Swen Page
From mispar
| Category | Category | Comment | Link | Annotated text |
|---|---|---|---|---|
| angle/acute angle | ח.ד.ד./חד | term | משנת_המדות#Fhur | חדות |
| angle/acute angle | ח.ד.ד./חד | term | ספר_יסודי_התבונה_ומגדל_האמונה#ohTc | זוית חדה |
| angle/acute angle | ח.ד.ד./חד | definition | ספר_יסודי_התבונה_ומגדל_האמונה#7qMI | the acute angle is less than the right [angle] in its value והזוית החדה היא המחסרת בערכה מן הנצבת |
| angle/acute angle | צ.ר.ר./צר | term | משנת_המדות#327H | זויות צרים |
| angle/acute angle | ח.ד.ד./חד | definition | ספר_היסודות_לאקלידס#V5db | The smaller than a right angle is called an '''acute angle'''. ואשר היא קטנה מנצבת תקרא חדה |
| acute angle/acute-angled | ח.ד.ד./חד | triangle | משנת_המדות#Kt5x | חדה |
| acute angle/acute-angled | ח.ד.ד./מחודד | triangle | ספר_יסודי_התבונה_ומגדל_האמונה#JbzH | המשלש מחדד הזויות |
| acute angle/acute-angled | ח.ד.ד./מחודד | triangle-definition | ספר_יסודי_התבונה_ומגדל_האמונה#lhjk | the first is that whose each angle is acute angle - this is called an acute-angled [triangle]. האחד הוא אשר כל זוית מזויותיו זוית חדה והוא הנקרא מחודד הזוית |
| acute angle/acute-angled | ח.ד.ד./חד | triangle-definition | ספר_היסודות_לאקלידס#pQ2M | The '''acute-angled triangle''' is that whose three angles are acute. ומשולש חד הזויות והוא אשר כל אחת מזויותיו השלש חדה |
| geometrical shape/angle | זוית | term | מלאכת_המספר#2ohU | זוית |
| geometrical shape/angle | זוית | term | מלאכת_המספר#fvYc | זויות |
| angle/angle between straight lines | י.ש.ר./ישרה | term | ספר_יסודי_התבונה_ומגדל_האמונה#IAoP | הזויות הישרות |
| angle/angle between straight lines | י.ש.ר./ישרה | term | ספר_יסודי_התבונה_ומגדל_האמונה#q7XH | הזוית הישרה |
| point/apex | ר.א.ש./ראש | term | משנת_המדות#jOb1 | ראש |
| line/apotome | ב.ד.ל./נבדל | definition | ספר_היסודות_לאקלידס#9THR | When a segment measurable in a square is subtracted from a straight line and the two lines are commensurable in square only, then the remaining straight line is unmeasurable; let it be called an apotome. ע כאשר הובדל מקו ישר מדבר בכח והיו השני קוים בכח לבד משותפים הנה הקו הנשאר בלתי מדבר ויקרא הנבדל |
| circle/arc | ק.ש.ת./קשת | term | משנת_המדות#dLJu | קשת |
| circle/arc | term | ספר_המספר_/_אברהם_אבן_עזרא#Gr5j | קשתות | |
| circle/arc | ק.ש.ת./קשת | definition | משנת_המדות#t84r | What is the arc? it is the part of the circle, as it is said: ''like the appearance of the rainbow that is in the cloud'' [Ezekiel 1, 28]. איזו היא קשת החלק מן העגול שנ' ''כמראה הקשת אשר יהיה בענן''יחזקאל א, כח |
| magnitude/area | ש.ב.ר./תשבורת | term | קצור_המספר#6eNb | תשבורת |
| magnitude/area | term | ספר_המספר_/_אברהם_אבן_עזרא#nSsY | תשבורת | |
| magnitude/area | מ.ש.ח./משיחה | term | משנת_המדות#hZNE | משיחה |
| magnitude/area | ר.ח.ב./רוחב | term | ספר_ג'יבלי_אלמוקבאלא#n6dR | רחב השטח |
| magnitude/area | ר.ב.ע./רבוע | term | ספר_הכללים_במספר#9C3m | רבוע |
| magnitude/area | ש.ב.ר./תשבורת | term | ספר_הכללים_במספר#pgiu | תשבורת |
| magnitude/area | ר.ב.ע./רבוע | term | ספר_הכללים_במספר#ez7p | רבוע |
| magnitude/area | ש.ב.ר./תשבורת | term | קצור_המספר#ZrPN | תשבורת |
| magnitude/area | ש.ט.ח./שטח | term | ספר_ג'יבלי_אלמוקבאלא#Q9z1 | שטחו |
| magnitude/area | ש.ט.ח./שטח | term | חשבון_השטחים#Fzc9 | שטח |
| magnitude/area | ש.ט.ח./שטח | term | תחבולות_המספר#F1Td | שטח |
| magnitude/area | מ.ש.ח./משיחה | term | ספר_הכללים_במספר#DJo0 | משיחת ה |
| magnitude/area | מ.ש.ח./משיחה | term | ספר_הכללים_במספר#INwY | משיחת ה |
| magnitude/area | ש.ב.ר./שבר | term | ספר_הכללים_במספר#1sqc | מידת שבריו |
| magnitude/area | ש.ב.ר./שבר | term | ספר_הכללים_במספר#lZxh | שבר |
| magnitude/area | ש.ב.ר./תשבורת | term | ספר_הכללים_במספר#a5B3 | תשברתו |
| magnitude/area | ש.ב.ר./תשבורת | term | ספר_האלזיברא#QTLl | תשבורתו |
| magnitude/area | ש.ב.ר./שברים | term | ספר_האלזיברא#Empf | שבריו |
| magnitude/area | ש.ב.ר./שברים | term | ספר_האלזיברא#XLwt | מספר שברי |
| magnitude/area | ש.ט.ח./שטח | term | ספר_ג'יבלי_אלמוקבאלא#s6dl | שטח |
| magnitude/area | term | ספר_המספר_/_אברהם_אבן_עזרא#bkNT | שברים | |
| sphere/axis | ב.ר.ח./בריח | definition | ספר_יסודי_התבונה_ומגדל_האמונה#d6V1 | if the sphere revolves around this diameter, we call it then the axis of the sphere. ואם יהיה הכדור סובב על האלכסון הזה אנו קורין לו בעת ההיא בריח הכדור |
| geometrical shape/base | י.ש.ב./תושבת | term | ספר_המספר_/_אליהו_מזרחי#FVdw | תושבתה |
| geometrical shape/base | ק.ב.ע./קבע | term | משנת_המדות#zDsv | קבע |
| geometrical shape/base | ק.ב.ע./קבע | definition | משנת_המדות#axVO | The base is that upon which two sides are fixed, ''upon which the house rests'' [Judges 16, 26; 29]. והקבע זה ששני צלעים קבועים עליו שני ''אשר הבית נכון עליהם''שופטים טז, כ"ו; כ"ט |
| geometrical shape/base | י.ש.ב./תושבת | term | מלאכת_המספר#ojMz | תושבת |
| geometrical shape/base | סוף | term | משנת_המדות#WWja | סוף |
| solid/beam | אריחי | term | האריתמטיקה_של_ניקומכוס#fZcR | מוגשמים אריחיים |
| point/center | צ.י.ר./ציר | center of a circle | ספר_יסודי_התבונה_ומגדל_האמונה#Xrf7 | ציר העגול |
| point/center | ר.כ.ז./מרכז | center of a circle | ספר_היסודות_לאקלידס#C6ny | מרכז העגולה |
| point/center | ר.כ.ז./מרכז | center of a circle | קצת_מענייני_חכמת_המספר#gyB1 | מרכז העגולה |
| point/center | צ.י.ר./ציר | term | ספר_הכללים_במספר#0qCr | ציר העגולה |
| point/center | צ.י.ר./ציר | term | ספר_הכללים_במספר#a6OG | ציר העגול |
| point/center | צ.י.ר./ציר | center of a sphere | ספר_יסודי_התבונה_ומגדל_האמונה#uFQI | ציר הכדור |
| point/center | ר.כ.ז./מרכז | term | Arithmetic_Textbook_by_R._Aharon_ben_Isaac#rFFw | מרכז |
| circle/chord | term | ספר_המספר_/_אברהם_אבן_עזרא#nGjl | יתר | |
| circle/chord | י.ת.ר./יתר | definition | משנת_המדות#ChKs | The chord is the holder of the arc, as it is said: ''bent bow'' [Isaiah 21, 15]. והיתר זה המחזיק בפי הקשת שנ' ''קשת דרוכה''ישעיה כא, טו |
| circle/chord | term | ספר_המספר_/_אברהם_אבן_עזרא#BVOw | יתרים | |
| circle/chord | י.ת.ר./יתר | term | משנת_המדות#ZqPD | יתר |
| circle/chord | י.ת.ר./מיתר | term | ספר_היסודות_לאקלידס#iAOK | מיתר |
| circle/chord | י.ת.ר./מיתר | term | ספר_היסודות_לאקלידס#NCXo | מיתר |
| surface/circle | ע.ג.ל./עגולה | term | אגרת_המספר#CEIL | עגולה |
| surface/circle | definition, #עגולה | ספר_יסודי_התבונה_ומגדל_האמונה#Tyz7 | we call the surface that the one line that surrounds it is circular, a circle if it is on a plane surface. והשטח אשר קו האחד המקיפו הוא עגול אנו קורין לשטח עגולה ישרה אם הוא בשטח ישר | |
| surface/circle | ע.ג.ל./עגולה | definition | ספר_היסודות_לאקלידס#uqaX | The '''circle''' is a plane figure contained by one line, within which there is one point such that all the straight lines drawn from it and terminated in the circumference are equal to one another. והעגולה היא תמונה פשוטה שוה יקיף אותה קו אחד בתוכה נקודה כל הקוים הישרים היוצאים ממנה ויכלו אל הקו ההוא שוים קצתם אל קצתם |
| surface/circle | ע.ג.ל./עגול | definition | ספר_יסודי_התבונה_ומגדל_האמונה#x37u | the circle is that which at its center there is one point such that all the lines that are drawn from it to the circumference are equal to each other in their value. השטח העגול הוא הנמצא באמצעיתו נקודה אחת אשר כל קו שתוציא ממנה אל הקו המקיף יהיו שוים בערכם זה לזה |
| surface/circle | ע.ג.ל./עגולה | term | מלאכת_המספר#yHjG | עגולה |
| surface/circle | term | ספר_המספר_/_אברהם_אבן_עזרא#oaVK | עגול | |
| surface/circle | term | ספר_המספר_/_אברהם_אבן_עזרא#Twc4 | עגולה | |
| surface/circle | ע.ג.ל./עגול | term | משנת_המדות#D5gU | עגול |
| surface/circle | term | ספר_המספר_/_אברהם_אבן_עזרא#PTuV | עגול | |
| surface/circle | ש.פ.ל./שפל | term | משנת_המדות#FIGD | שפלה |
| surface/circle | ע.ג.ל./עגולה | term | משנת_המדות#43ng | עגלה |
| line/circular line | ע.ג.ל./עגול | definition | ספר_יסודי_התבונה_ומגדל_האמונה#NCuE | The circular line is the one on which you cannot find three points that are in the same direction, and inside of it there is a point that is equidistant from all the points of the circle. והקו העגול הוא כל אשר אי אתה יכול למצוא ג' נקדות על נכח וימצא בתוכו נקדה שיהיה מרחקה מכל נקדות העגול מרחק אחד |
| line/circular line | ע.ג.ל./עגול | term | ספר_יסודי_התבונה_ומגדל_האמונה#bObt | קו עגול |
| geometric relations/commensurable | ש.ת.פ./משותף | definition | ספר_היסודות_לאקלידס#qiVv | Those that have magnitudes, as lines, surfaces, and solids, that are said to be '''commensurable''', are those that are measured by the same measure.
בעלי השעורים מן הקוים והשטחים והמוגשמים אשר יאמר להם |
| geometric relations/commensurable | ש.ת.פ./בלתי משותפים | definition-incommensurable | ספר_היסודות_לאקלידס#lGqt | Those that are said to be '''incommensurable''' are those that cannot be measured the same measure. ואשר יאמר להם בלתי משותפים הם אשר לא ישער אותם כלם שעור אחד |
| geometric relations/commensurable in square | ש.ת.פ./בלתי משותפים בכח | definition-incommensurable in square | ספר_היסודות_לאקלידס#zQYu | They are said to be '''incommensurable in square''', when the squares [that are generated] from them cannot be measured by the same area.
ויאמר להם בלתי משותפים בכח כאשר לא יהיה למרובעים |
| geometric relations/commensurable in square | ש.ת.פ./משותפים בכח | definition | ספר_היסודות_לאקלידס#shEk | Straight lines are said to be '''commensurable in square''', when the squares that are generated from them are measured by the same area. והקוים הישרים אשר יאמר להם המשותפים בכח כאשר היה למרובעים ההוים מהם שטח ישער אותם |
| surface/concave surface | ע.ק.מ./עקמומי | definition | ספר_יסודי_התבונה_ומגדל_האמונה#Rmia | the concave surface is the plane that encompasses the dome from beneath; it is also either circular or curved. והפרוש העקמומי הוא הרקיע המקיף את גב הקובה מלמטה ויהיה כמו כן עגול או עקום |
| surface/concave surface | ע.ק.מ./עקמומי | term | ספר_יסודי_התבונה_ומגדל_האמונה#Ue2N | עקמומי |
| solid/cone | אלון | term | ספר_יסודי_התבונה_ומגדל_האמונה#7Wx1 | אלון |
| solid/cone | אתרוג | term | משנת_המדות#8LjV | אתרוג |
| solid/cone | מ.ש.כ./משוך | definition | משנת_המדות#6tE5 | The conical, whose apex is sharp and base is planar, whether quadrilateral, or circular, or triangular: ז' והמשוך ראשו חד וסופו {{#annot:term|588,2554|epCp}}ממוצע{{#annotend:epCp}} ואפי' מרובע או שיהיה עגול או משולש |
| solid/cone | אלוני | term | ספר_יסודי_התבונה_ומגדל_האמונה#w4jz | הגולם האלוני |
| solid/cone | ח.ר.ט./חרוט | term | ספר_יסודי_התבונה_ומגדל_האמונה#eKVd | חרוטות |
| solid/cone | ח.ר.ט./חרוט | term | ספר_יסודי_התבונה_ומגדל_האמונה#PHTK | הגולם החרוט |
| solid/cone | אלוני | term | ספר_יסודי_התבונה_ומגדל_האמונה#NCUz | האלוני |
| geometric relations/congruent figures | ש.ו.ה./שוה | solids-definition | ספר_היסודות_לאקלידס#68bD | The equal similar solid shapes are those that each solid of which is contained by the same number of surfaces as the number of the surfaces containing the other, and each surface is similar and equal in measure to its corresponding surface in the other solid. התמונות המוגשמות השוות הדומות הם אשר יקיפו בכל מוגשם מהם ממנין השטחים כמו מנין מה שיקיפו באחר ויהיה כל שטח מאחד מהם דומה ושוה השיעור לשטח אשר הוא גילו מן המוגשם האחר ועל בריאתו |
| surface/convex surface | ק.ב.ב./מקובה | term | משנת_המדות#nssa | מקובה |
| surface/convex surface | ק.ב.ב./קבוב | definition | ספר_יסודי_התבונה_ומגדל_האמונה#Q7bh | the convex surface is the plane that encompasses the dome from above; this surface is either circular or curved. והפרוש הקבוב הוא הרקיע המקיף את קו הקובה מלמעלה והרקיע הזה יהיה עגול ויהיה עקום |
| surface/convex surface | ק.ב.ב./קובה | term | משנת_המדות#8Lrz | קובה |
| surface/convex surface | ק.ב.ב./קבוב | term | ספר_יסודי_התבונה_ומגדל_האמונה#txDc | קבוב |
| solid/cube | ע.ק.ב./מעוקב | term | בר_נותן_טעם#n3HE | גשם מעוקב |
| solid/cube | ע.ק.ב./מעוקב | term | Arithmetic_Textbook_by_R._Aharon_ben_Isaac#gzFs | מעוקב |
| line/curve | ע.ק.מ./עקום | term | ספר_יסודי_התבונה_ומגדל_האמונה#MU5o | קו עקום |
| line/curve | ע.ק.מ./עקום | definition | ספר_יסודי_התבונה_ומגדל_האמונה#q5Cb | The curve is any line on which you cannot find three points that are in the same direction and you cannot find also any point in it that is equidistant from all the points of the curve. והקו העקום כל קו שאי אתה מוצא ג' נקדות על נכח אחד ואף נקדה שיהיה מרחקה מנקדות העקום מרחק אחד אי אתה מוצא בו |
| solid/cylinder | ע.ג.ל./עגולה | term | ספר_הכללים_במספר#Mt2g | מצבה עגולה |
| solid/cylinder | אצטוונא | term | ספר_המספר_/_אליהו_מזרחי#Ensz | האצטוונא העגולה |
| solid/cylinder | ע.ג.ל./עגולה | term | ספר_הכללים_במספר#zGsp | מצבה עגולה |
| solid/cylinder | אצטוונא | term | ספר_המספר_/_אליהו_מזרחי#gbMU | איצטיונא עגולה |
| solid/cylinder | אצטוונא | term | ספר_המספר_/_אליהו_מזרחי#rax7 | אצטוונא |
| linear dimension/depth | ע.מ.ק./עומק | term | מלאכת_המספר#i136 | עומק |
| linear dimension/depth | ע.מ.ק./עומק | term | מלאכת_המספר#SFsc | עומק |
| line/diagonal | אלכסון | term | ספר_יסודי_התבונה_ומגדל_האמונה#mxol | קו האלכסון |
| line/diagonal | אלכסון | term | ספר_הכללים_במספר#HaZi | אלכסון |
| line/diagonal | אלכסון | term | ספר_הכללים_במספר#VGc4 | אלכסון |
| line/diagonal | חוט | definition | משנת_המדות#1yoc | The diagonal is the cutting from an angle to an angle, end to end, and it is the greater length of the surface. והחוט זה המפסיק מזוית לזוית מן הקצה אל הקצה והוא היותר בארכו של גג |
| line/diagonal | חוט | term | משנת_המדות#gn1H | חוט |
| line/diameter | ק.ט.ר./קוטר | term | ספר_המספר_/_אליהו_מזרחי#wKt6 | קוטר |
| line/diameter | אלכסון | diameter of a sphere-definition | ספר_יסודי_התבונה_ומגדל_האמונה#ZeeF | every line passing through the center of the sphere and reaching at its both sides to the surface is called the diameter of the sphere. וכל קו העובר על ציר הכדור והוא מגיע בשני צדיו אל הפרוש נקרא אלכסון הכדור |
| line/diameter | אלכסון | term | ספר_הכללים_במספר#vRfG | אלכסונו |
| line/diameter | אלכסון | diameter of a circle-definition | ספר_יסודי_התבונה_ומגדל_האמונה#WT1p | every line passing through the center of the circle and reaching at its both sides to the circumference is called the diameter of the circle. וכל קו אשר הוא עובר על ציר העגול ומגיע מב' פנותיו אל הקו המקיף הוא נקרא אלכסון העגול |
| line/diameter | אלכסון | term | מלאכת_המספר#kR8y | אלכסון |
| line/diameter | חוט | term | משנת_המדות#6q7O | חוט |
| line/diameter | term | ספר_המספר_/_אברהם_אבן_עזרא#Ez31 | אלכסון | |
| line/diameter | ק.ט.ר./קוטר | definition | ספר_היסודות_לאקלידס#v3Ba | The '''diameter''' of the circle is any straight line, drawn through the center of the circle and terminated in both directions by its circumference, that bisects [the circle]. וקוטר העגולה הוא קו ישר ילך במרכז העגולה ויכלה בשני הצדדי' אל הקו המקיף אותה והוא יחתכנה בשני חצאים |
| magnitude/distance | ר.ח.ק./רוחק | term | מלאכת_המספר#BkNI | רוחק |
| Elements/Elements II-1 | a·∑bᵢ=∑(a·bᵢ) | ספר_המספר_/_אליהו_מזרחי#me96 | For every number divided into many parts randomly, the number that is generated from the product of a number by the whole given divided number is equal to the number generated from the sum of the products of that number by each part of the divided number.
: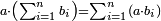 השנית שכל מספר נחלק לחלקים רבים איך מה שקרה הנה המספר ההווה מהכאת מספר מה עם המספר המונח הנחלק בכללו הוא שוה למספר ההווה מהכאת המספר ההוא עם כל אחד מחלקי המספר הנחלק כאשר יקובצו
השנית שכל מספר נחלק לחלקים רבים איך מה שקרה הנה המספר ההווה מהכאת מספר מה עם המספר המונח הנחלק בכללו הוא שוה למספר ההווה מהכאת המספר ההוא עם כל אחד מחלקי המספר הנחלק כאשר יקובצו | |
| Elements/Elements II-1 | a·∑bᵢ=∑(a·bᵢ) | ספר_מעשה_חושב#4J1r | When there are two given numbers and one of them is divided into parts, as many as they may be, the product of the first number by the second is equal to the [sum of] the products of each of the parts of the first number by the second.
: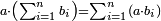 ב כאשר היו שני מספרים מונחים וחולק המספר האחד לחלקים כמה שיהיו הנה שטח המספר האחד בשני שוה לשטחי כל אחד מחלקי המספר האחד בשני מקובצים
ב כאשר היו שני מספרים מונחים וחולק המספר האחד לחלקים כמה שיהיו הנה שטח המספר האחד בשני שוה לשטחי כל אחד מחלקי המספר האחד בשני מקובצים | |
| Elements/Elements II-1 | a·∑bᵢ=∑(a·bᵢ) | לקוטים_מספר_פראלוקא#t9Uk | If you have two numbers and you divide one of the two numbers, then you multiply each part by the second number, the total sum is the same as the product of the first number by the second.
: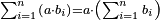 156 אם יש לך ב' מספרים ותחלק א' מהב' מספרים ותכפול כל חלק על המספר השני הנה הסך העולה הוא כמו מה שיעלה מכפל המספר הראשון על השני
156 אם יש לך ב' מספרים ותחלק א' מהב' מספרים ותכפול כל חלק על המספר השני הנה הסך העולה הוא כמו מה שיעלה מכפל המספר הראשון על השני | |
| Elements/Elements II-1 | ספר_החשבון_והמדות#fGS3 | I say: if you divide any number into parts as you wish, [the sum of] the products of each of the parts by the whole number is equal to the square of the whole number.
:![\scriptstyle{\color{OliveGreen}{\sum_{k=1}^n \left[\left(\sum_{i=1}^n a_i\right)\sdot a_k\right]=\left(\sum_{i=1}^n a_i\right)^2}}](/mediawiki/images/math/9/9/f/99fd3737e42a1acf970a92fb7a977c13.png) ואומר כל מספר שחלקת אותו לחלקים איך שרצית הנה כפל כל אחד מהחלקים על כל המספר מקובץ שוה למרובע כל המספר
ואומר כל מספר שחלקת אותו לחלקים איך שרצית הנה כפל כל אחד מהחלקים על כל המספר מקובץ שוה למרובע כל המספר | ||
| Elements/Elements II-1 | a·∑bᵢ=∑(a·bᵢ) | ספר_המלכים#Sjh3 | For every two numbers, such that one of them is divided into as many parts as there are, [the product of] the number that is not divided by the divided number is equal to the sum of its products by each part of the divided number.
: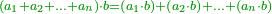 כל שני מספרים יחלק אחד מהם בחלקים כמו שיהיו הנה המספר שלא חולק במספר שחולק כמו הכאתו בכל חלקי המספר הנחלק כאשר יקובצו
כל שני מספרים יחלק אחד מהם בחלקים כמו שיהיו הנה המספר שלא חולק במספר שחולק כמו הכאתו בכל חלקי המספר הנחלק כאשר יקובצו | |
| Elements/Elements II-1 | definition | ספר_המספר_/_אליהו_מזרחי#8mWV | It was already clarified in Euclid's Book of Elements, in the [second] section, in the first proposition that for any two straight lines, one of which is cut into segments as many as they may be, the sum of the surfaces generated from the whole straight line and each of the segments of the other straight line equals the surface generated from the whole straight line and the whole divided line.
: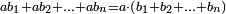 וזה שכבר התבאר בספר היסודות לאקלידס במאמר השלישי ממנו בתמונה הראשונה שכל שני קוים שנחלק אחד מהם לחלקים כמה שיהיו הנה השטח ההוא מהקו האחד כלו עם כל אחד מחלקי הקו האחר יחד הוא שוה לשטח ההווה מהקו האחד עם כל הקו הנחלק
וזה שכבר התבאר בספר היסודות לאקלידס במאמר השלישי ממנו בתמונה הראשונה שכל שני קוים שנחלק אחד מהם לחלקים כמה שיהיו הנה השטח ההוא מהקו האחד כלו עם כל אחד מחלקי הקו האחר יחד הוא שוה לשטח ההווה מהקו האחד עם כל הקו הנחלק | |
| Elements/Elements II-1 | 10,2+3+5+2 | לקוטים_מספר_פראלוקא#1Q9z | Example: the two numbers are 10 and 12 and we divide 12 to 2, 3, 5, 2, so their sum is 12. המשל הנה ב' מספרים והם י' וי"ב ונחלק י"ב על ב' ועל ג' ועל ה' ועל ב' והנה כלם י"ב | |
| Elements/Elements II-1 | 4,2+3+5 | ספר_המספר_/_אליהו_מזרחי#1rE2 | Example: if we divide the 10 into three parts randomly, one part of them is 2, the second is 3 and the third is 5. משל זה אם נחלק הי' לג' חלקים איך מה שקרה והיה החלק האחד מהם מספר ב' והשני מספר ג' והשלישי מספר ה' | |
| Elements/Elements II-10 | 12,8 | לקוטים_מספר_פראלוקא#wuja | Example: we have 12, you divide it to 6 and 6, then add to 12 another number. Suppose that we add 8 to it. המשל יש לנו מספר י"ב ותחלקהו על ו'ו' ותוסיף על י"ב 148vמספר אחר ונניח כי נוסיף בם ח' | |
| Elements/Elements II-10 | (a+b)²+b²=2·((½a)²+((½a)+b)²) | ספר_המלכים#CVU6 | For any even number divided into half and another number is added to it, [the sum of] twice the product of half the number by itself and twice the product of half the number plus the additional [number] by itself is equal to [the sum of] the product of the [whole] number plus the additional [number] by itself and [the product] of the additional [number] by itself.
:![\scriptstyle{\color{OliveGreen}{\left[2\sdot\left(\frac{1}{2}\sdot a\right)^2\right]+\left[2\sdot\left[\left(\frac{1}{2}\sdot a\right)+b\right]^2\right]=\left(a+b\right)^2+b^2}}](/mediawiki/images/math/b/6/e/b6e91cc251302ce6002e5d5c009f9286.png) כל מספר זוג יחלק לחציים ונוסף בו מספר אחר הנה ההווה מהכאת חצי המספר בכמהו שני פעמים והכאת חצי המספר עם התוספת בכמהו שני פעמים
כל מספר זוג יחלק לחציים ונוסף בו מספר אחר הנה ההווה מהכאת חצי המספר בכמהו שני פעמים והכאת חצי המספר עם התוספת בכמהו שני פעמים | |
| Elements/Elements II-10 | (a+b)²+b²=2·((½a)²+((½a)+b)²) | ספר_החשבון_והמדות#w9pW | If you divide any number into half and add to it another number, [the sum of] the square of the whole number plus the additional [number] and the square of the additional [number] is equal to twice [the sum of] the square of half the number and the square of half the number plus the additional [number] together.
:![\scriptstyle{\color{OliveGreen}{\left(a+b\right)^2+b^2=2\sdot\left[\left(\frac{1}{2}\sdot a\right)^2+\left[\left(\frac{1}{2}\sdot a\right)+b\right]^2\right]}}](/mediawiki/images/math/0/1/f/01f5c12dc0db0f20f362991f00bed1d8.png) עוד כל מספר שחלקת אותו לשני חציים והוספת עליו מספר אחר הנה מרובע המספר עם התוספת יחד ומרובע התוספת בעצמו הם כפל שני המרובעים שהם מרובע חצי המספר ומרובע חצי המספר עם התוספת יחד כאשר {{#annot:term|178,2083|Ycx9}}יחוברו{{#annotend:Ycx9}}
עוד כל מספר שחלקת אותו לשני חציים והוספת עליו מספר אחר הנה מרובע המספר עם התוספת יחד ומרובע התוספת בעצמו הם כפל שני המרובעים שהם מרובע חצי המספר ומרובע חצי המספר עם התוספת יחד כאשר {{#annot:term|178,2083|Ycx9}}יחוברו{{#annotend:Ycx9}} | |
| Elements/Elements II-10 | 10,2 | ספר_המספר_/_אליהו_מזרחי#0WFg | Example: we have the number ten, we divide it into two halves and add to it the number 2, so it bacomes 12. דמיון יש לנו מספר עשרה וחלקנוהו לשני חציים והוספנו עליו מספר ב' ונהיה י"ב | |
| Elements/Elements II-10 | (a+b)²+b²=2·((½a)²+((½a)+b)²) | ספר_המספר_/_אליהו_מזרחי#FJSe | Any number that you divide into half and add to it another number, [the sum of] the square of the [whole] number plus the additional [number] and the square of the additional [number] is equal to twice [the sum of] the square of half the number and the square of half the number plus the additional [number] when they are summed together.
:![\scriptstyle{\color{OliveGreen}{\left(a+b\right)^2+b^2=2\sdot\left[\left(\frac{1}{2}\sdot a\right)^2+\left[\left(\frac{1}{2}\sdot a\right)+b\right]^2\right]}}](/mediawiki/images/math/0/1/f/01f5c12dc0db0f20f362991f00bed1d8.png) עוד כל מספר שחלקת אותו לשני חצאים והוספת עליו מספר אחר הנה מרובע המספר עם התוספת יחד ומרובע התוספת בעצמו הם כפל שני המרובעים שהם מרובע חצי המספר ומרובע חצי המספר עם התוספת יחד כאשר יחוברו
עוד כל מספר שחלקת אותו לשני חצאים והוספת עליו מספר אחר הנה מרובע המספר עם התוספת יחד ומרובע התוספת בעצמו הם כפל שני המרובעים שהם מרובע חצי המספר ומרובע חצי המספר עם התוספת יחד כאשר יחוברו | |
| Elements/Elements II-10 | (a+b)²+b²=2·((½a)²+((½a)+b)²) | לקוטים_מספר_פראלוקא#ijBn | If you divide any number into two equal parts, then add another number to the divided number, square it and add to it the square of the [number] you added, it is twice [the sum of] the square of the additional [number] plus half [the number] with the square of half [the number].
:![\scriptstyle\left(a+b\right)^2+b^2=2\sdot\left[\left[\left(\frac{1}{2}\sdot a\right)+b\right]^2+\left(\frac{1}{2}\sdot a\right)^2\right]](/mediawiki/images/math/5/e/9/5e9b4c74db157126a2fd01aad4693992.png) 165 אם תחלק איזה מספר על ב' חלקים שוים ואחר תוסיף מספר אחר על המספר המחולק ותרבענו ותוסיף על זה מרובע שהוספת יהיה ב' פעמים כמו כפל ממה שיעלה התוספת הנוסף על החצי עם מרובע החצי נוספים
165 אם תחלק איזה מספר על ב' חלקים שוים ואחר תוסיף מספר אחר על המספר המחולק ותרבענו ותוסיף על זה מרובע שהוספת יהיה ב' פעמים כמו כפל ממה שיעלה התוספת הנוסף על החצי עם מרובע החצי נוספים | |
| Elements/Elements II-10 | 10,2 | ספר_החשבון_והמדות#zIUc | Example: we have the number ten, we divide it into two halves, which are 5, and add to it another number 2, so it bacomes 12. דמיון יש לנו מספר מניינו עשרה וחלקנוהו לשני חצאים על ה' והוספנו עליו 33rמספר אחר ב' ונהיה י"ב | |
| Elements/Elements II-2 | 12,3+4+5 | לקוטים_מספר_פראלוקא#Gj4L | Example: we wish to divide 12 into three parts 5, 3, 4. המשל נרצה לעשות מי"ב ג' חלקים ה' ג' ד' | |
| Elements/Elements II-2 | ∑ₖ((∑ᵢaᵢ)·aₖ)=(∑ᵢaᵢ)² | ספר_החשבון_והמדות#DxvK | For every number that you divide randomly into two parts, the sum of the products of each of the two parts by the whole number is equal to the square of the whole number.
:![\scriptstyle{\color{OliveGreen}{\left[\left(a+b\right)\sdot a\right]+\left[\left(a+b\right)\sdot b\right]=\left(a+b\right)^2}}](/mediawiki/images/math/8/a/a/8aae9db1c4694d4c859578e458487970.png) עוד כל מספר שחלקת אותו לשני חלקים איך שקרה הנה כפל כל אחד משני החלקים על כל המספר מקובץ שוה למרובע כל המספר
עוד כל מספר שחלקת אותו לשני חלקים איך שקרה הנה כפל כל אחד משני החלקים על כל המספר מקובץ שוה למרובע כל המספר | |
| Elements/Elements II-2 | 10,7+3 | ספר_החשבון_והמדות#KF6o | Example: the number ten; we divide it into 7 and 3. דמיון המספר עשרה וחלקנוהו על ז' וג' | |
| Elements/Elements II-2 | ∑ₖ((∑ᵢaᵢ)·aₖ)=(∑ᵢaᵢ)² | קצת_מענייני_חכמת_המספר#4EHv | If you divide any number into parts as you wish, [the sum of] the products of each of the parts by the whole number is equal to the square of the whole number.
:![\scriptstyle{\color{OliveGreen}{\sum_{k=1}^n \left[\left(\sum_{i=1}^n a_i\right)\sdot a_k\right]=\left(\sum_{i=1}^n a_i\right)^2}}](/mediawiki/images/math/9/9/f/99fd3737e42a1acf970a92fb7a977c13.png) אחת כל מספר שחלקת אותו לחלקים איך שרצית הנה הכאת כל אח' מהחלקי' בכל המספר השוה למרובע הכל
אחת כל מספר שחלקת אותו לחלקים איך שרצית הנה הכאת כל אח' מהחלקי' בכל המספר השוה למרובע הכל | |
| Elements/Elements II-2 | ∑ₖ((∑ᵢaᵢ)·aₖ)=(∑ᵢaᵢ)² | לקוטים_מספר_פראלוקא#nMHc | If you have a number and you divide iy into parts as you wish, if you multiply each part by the divided number, then sum all the [products] they are equal to the divided number multiplied by itself.
:![\scriptstyle\sum_{k=1}^n \left[\left(\sum_{i=1}^n a_i\right)\sdot a_k\right]=\left(\sum_{i=1}^n a_i\right)^2](/mediawiki/images/math/2/a/1/2a190390030d9c138d548197fab10837.png) 157 אם יש לך מספר אחד ותחלק אותו לכ"כ חלקים שתרצה אם תכפול כל חלק על המספר המחולק ותקבץ כל החלקים יהיו שוים אל המספר המחולק כפול על עצמו
157 אם יש לך מספר אחד ותחלק אותו לכ"כ חלקים שתרצה אם תכפול כל חלק על המספר המחולק ותקבץ כל החלקים יהיו שוים אל המספר המחולק כפול על עצמו | |
| Elements/Elements II-2 | 12,3+4+5 | ספר_החשבון_והמדות#pdf0 | Example: the number 12; we divide it into three, four and five. דמיון המספר י"ב וחלקנוהו על שלשה וארבעה וחמשה | |
| Elements/Elements II-2 | ∑ₖ((∑ᵢaᵢ)·aₖ)=(∑ᵢaᵢ)² | ספר_המספר_/_אליהו_מזרחי#mQeQ | Any number that you divide into parts as you wish, [the sum of] the products of each of the parts by the whole number is equal to the square of the whole number.
:![\scriptstyle{\color{OliveGreen}{\sum_{k=1}^n \left[\left(\sum_{i=1}^n a_i\right)\sdot a_k\right]=\left(\sum_{i=1}^n a_i\right)^2}}](/mediawiki/images/math/9/9/f/99fd3737e42a1acf970a92fb7a977c13.png) והוא שכל מספר שחלקת אותו לחלקים איך שרצית הנה הכאת כל אחד מהחלקים עם כל המספר שוה למרובע כל המספר
והוא שכל מספר שחלקת אותו לחלקים איך שרצית הנה הכאת כל אחד מהחלקים עם כל המספר שוה למרובע כל המספר | |
| Elements/Elements II-2 | 12,3+4+5 | ספר_המספר_/_אליהו_מזרחי#Nxlm | Example: the number 12, we divide it to three, four and five. דמיון זה המספר י"ב וחלקנוהו על שלשה וארבעה וחמשה | |
| Elements/Elements II-3 | (a+b)·b=(a·b)+b² | לקוטים_מספר_פראלוקא#fYtF | If you divide any number into two parts, then multiply any of the two parts by the divided number and keep the product, so will be the result if you multiply the same part by itself, then add to it the product of the one part by the other.
: 158 אם תחלק איזה מספר על ב' חלקים ותכפול החלק שיהיה מב' החלקים על המספר המחולק ושמור העולה כך יעלה אם תכפול אותו חלק על עצמו ותוסיף בו העולה מהכפל מהחלק האחד על השני
158 אם תחלק איזה מספר על ב' חלקים ותכפול החלק שיהיה מב' החלקים על המספר המחולק ושמור העולה כך יעלה אם תכפול אותו חלק על עצמו ותוסיף בו העולה מהכפל מהחלק האחד על השני | |
| Elements/Elements II-3 | (a+b)·b=(a·b)+b² | קצת_מענייני_חכמת_המספר#PVyt | For any number divided into two parts as you wish, the product of the whole number by any of its two parts is equal to the product of the one part by the other plus the square of the part by which you multiplied the whole number.
: ב כל מספר שחלקת אותו לב' חלקים איך שקרה הנה הכאת המספר כלו עם כל אחד מב' חלקיו איזה שיהיה שוה להכאת החלק האחד עם האחר ולמרובע החלק אשר בו הכית כל המספר
ב כל מספר שחלקת אותו לב' חלקים איך שקרה הנה הכאת המספר כלו עם כל אחד מב' חלקיו איזה שיהיה שוה להכאת החלק האחד עם האחר ולמרובע החלק אשר בו הכית כל המספר | |
| Elements/Elements II-3 | 10,3+7 | קצת_מענייני_חכמת_המספר#MLKI | Example: the number 10 is divided into two parts - 7 and 3. כמשל מספר הי' נחלק לב' חלקים לז' ולג' | |
| Elements/Elements II-3 | (a+b)·b=(a·b)+b² | ספר_החשבון_והמדות#Eu5r | For any number divided into two parts as you wish, the product of the whole number by any of its two parts is equal to the product of the one part by the other plus the square of the part by which you multiplied the whole number.
: עוד כל מספר שחלקת אותו בשני חלקים איך שקרה הנה כפל המספר כולו על אחד משני חלקיו איזה שיהיה שוה לכפול החלק האחד על השני ולמרובע החלק משניהם אשר כפלת על כל המספר
עוד כל מספר שחלקת אותו בשני חלקים איך שקרה הנה כפל המספר כולו על אחד משני חלקיו איזה שיהיה שוה לכפול החלק האחד על השני ולמרובע החלק משניהם אשר כפלת על כל המספר | |
| Elements/Elements II-3 | 12,4+8 | לקוטים_מספר_פראלוקא#XIpE | Example: we have 12 and you divide it to 8 and 4. המשל אם יש לנו י"ב ותחלק אותו ח' ד' | |
| Elements/Elements II-3 | 10,3+7 | ספר_החשבון_והמדות#zmnp | Example: the number ten, we divide it into two parts - three and seven. דמיון המספר עשרה חלקנוהו לשני חלקים על שלשה ושבעה | |
| Elements/Elements II-3 | (a+b)·b=(a·b)+b² | ספר_מעשה_חושב#BvNU | When a number is divided into two parts, the product of the whole number by one of its parts is equal to the product of the one part by the other plus the square of the mentioned part.
: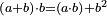 ד כאשר חולק מספר מה בשני חלקים הנה שטח כל המספר באחד מחלקיו שוה לשטח החלק האחד באחר ולמרובע החלק אשר זכרנו
ד כאשר חולק מספר מה בשני חלקים הנה שטח כל המספר באחד מחלקיו שוה לשטח החלק האחד באחר ולמרובע החלק אשר זכרנו | |
| Elements/Elements II-3 | (a+b)·b=(a·b)+b² | ספר_המספר_/_אליהו_מזרחי#oIDS | For any number that you divide into two parts randomly, the product of the whole number by any of its two parts is equal to the product of the one part by the other plus the square of the part by which you have multiplied the whole number.
: עוד כל מספר שחלקת אותו לשני חלקים איך שקרה הנה הכאת המספר כלו עם כל אחד משני חלקיו איזה שיהיה שוה להכאת החלק האחד עם השני ולמרובע החלק אשר הכית עם כל המספר
עוד כל מספר שחלקת אותו לשני חלקים איך שקרה הנה הכאת המספר כלו עם כל אחד משני חלקיו איזה שיהיה שוה להכאת החלק האחד עם השני ולמרובע החלק אשר הכית עם כל המספר | |
| Elements/Elements II-3 | 10,3+7 | ספר_המספר_/_אליהו_מזרחי#CANO | Example: the number ten, we divide into two parts - three and seven. דמיון המספר עשרה חלקנוהו לשני חלקים על שלשה ושבעה | |
| Elements/Elements II-4 | 10,3+7 | ספר_החשבון_והמדות#hh0J | Example: the number ten; we divide it into three and seven. דמיון המספר עשרה חלקנוה על שלשה ושבעה | |
| Elements/Elements II-4 | (a+b)²=a²+b²+2(a·b) | ספר_מעשה_חושב#lUMx | When a number is added to a number, the square of the two numbers that are summed together is equal to [the sum of] the squares of these numbers and twice the product of the one by the other.
:![\scriptstyle\left(a+b\right)^2=a^2+b^2+\left[2\sdot\left(a\sdot b\right)\right]](/mediawiki/images/math/7/2/7/727b0c35fd93a7a347bed8413905049f.png) ו כאשר נוסף על מספר מונח מספר מה הנה מרובע שני המספרים מחוברים שוה למרובעי המספרים ההם ולכפל שטח זה בזה
ו כאשר נוסף על מספר מונח מספר מה הנה מרובע שני המספרים מחוברים שוה למרובעי המספרים ההם ולכפל שטח זה בזה | |
| Elements/Elements II-4 | (a+b)²=a²+b²+2(a·b) | קצת_מענייני_חכמת_המספר#v7Dx | For any number divided into two parts as you wish, the square of the whole number is equal to [the sum of] the squares of the two parts and twice the product of the one part by the other.
:![\scriptstyle{\color{OliveGreen}{\left(a+b\right)^2=a^2+b^2+\left[2\sdot\left(a\sdot b\right)\right]}}](/mediawiki/images/math/d/f/2/df26d1cdaf50844081193e48666b1eeb.png) ג כל מספר שחלקת אותו לשני חלקים איך שקרה הנה מרובע כל המספר שוה לב' המרובעים ההווים מב' החלקים ולהכאת החלק האחד עם חבירו ב' פעמים
ג כל מספר שחלקת אותו לשני חלקים איך שקרה הנה מרובע כל המספר שוה לב' המרובעים ההווים מב' החלקים ולהכאת החלק האחד עם חבירו ב' פעמים | |
| Elements/Elements II-4 | (a+b)²=a²+b²+2(a·b) | ספר_המספר_/_אליהו_מזרחי#TApz | For any number divided into two parts randomly, the square of the whole number is equal to [the sum of] the squares of the two parts and twice the product of the one part by the other.
:![\scriptstyle\left(a+b\right)^2=a^2+b^2+\left[2\sdot\left(a\sdot b\right)\right]](/mediawiki/images/math/7/2/7/727b0c35fd93a7a347bed8413905049f.png) האחת שכל מספר נחלק לשנים חלקים איך מה שקרה הנה המרובע ההווה מן המספר כלו הוא שוה לשני המרובעים ההווים משני חלקיו עם כפל המספר ההווה מהכאת החלק האחד עם האחר
האחת שכל מספר נחלק לשנים חלקים איך מה שקרה הנה המרובע ההווה מן המספר כלו הוא שוה לשני המרובעים ההווים משני חלקיו עם כפל המספר ההווה מהכאת החלק האחד עם האחר | |
| Elements/Elements II-4 | 10,3+7 | קצת_מענייני_חכמת_המספר#OusC | Example: the number 10 is divided into 7 and 3. כמשל הי' נחלק לז' ולג' | |
| Elements/Elements II-4 | (a+b)²=a²+b²+2(a·b) | ספר_המספר_/_אליהו_מזרחי#oC2j | For any number that you divide into two parts randomly, the square of the whole number is equal to [the sum of] the squares of the two parts and twice the product of the one part by the other.
:![\scriptstyle{\color{OliveGreen}{\left(a+b\right)^2=a^2+b^2+\left[2\sdot\left(a\sdot b\right)\right]}}](/mediawiki/images/math/d/f/2/df26d1cdaf50844081193e48666b1eeb.png) עוד כל מספר שחלקת אותו לשני חלקים איך שקרה הנה מרובע כל המספר שוה לשני המרובעי' ההווים משני החלקים ולהכאת החלק האחד עם חברו פעמים
עוד כל מספר שחלקת אותו לשני חלקים איך שקרה הנה מרובע כל המספר שוה לשני המרובעי' ההווים משני החלקים ולהכאת החלק האחד עם חברו פעמים | |
| Elements/Elements II-4 | 10,3+7 | ספר_המספר_/_אליהו_מזרחי#6oZu | Example: if we divide the 10 into two parts randomly, one part of them is 3 and the other 7. משל זה אם נחלק הי' לשנים חלקים איך מה שקרה והיה החלק האחד מהם ג' והאחר ז' | |
| Elements/Elements II-4 | (a+b)²=a²+b²+2(a·b) | ספר_החשבון_והמדות#q5ib | For any number divided into two parts as you wish, the square of the whole number is equal to [the sum of] the squares of the two parts and twice the product of the one part by the other.
:![\scriptstyle{\color{OliveGreen}{\left(a+b\right)^2=a^2+b^2+\left[2\sdot\left(a\sdot b\right)\right]}}](/mediawiki/images/math/d/f/2/df26d1cdaf50844081193e48666b1eeb.png) עוד כל מספר שחלקת אותו לשני חלקים איך שקרה הנה מרובע כל המספר שוה לשני המרובעים ההוים משני החלקים ולכפל החלק האחד על חברו פעמים
עוד כל מספר שחלקת אותו לשני חלקים איך שקרה הנה מרובע כל המספר שוה לשני המרובעים ההוים משני החלקים ולכפל החלק האחד על חברו פעמים | |
| Elements/Elements II-4 | (a+b)²=a²+b²+2(a·b) | לקוטים_מספר_פראלוקא#lb40 | If you divide any number into two and multiply each part by itself, you multiply also one [part] by the other and multiply [the product] by 2, then you sum all, the result is equal to the divided number multiplied by itself.
:![\scriptstyle a^2+b^2+\left[2\sdot\left(a\sdot b\right)\right]=\left(a+b\right)^2](/mediawiki/images/math/8/2/e/82e411d047c5fc9961bdf7306ae1b255.png) 159 אם תחלק איזה מספר שיהיה על ב' ותכפול כל חלק על עצמו וג"כ כפול הא' על הב' וכפלם על ב' ותקבץ הכל הנה העולה יהיה שוה אל המספר המחולק כפול על עצמו
159 אם תחלק איזה מספר שיהיה על ב' ותכפול כל חלק על עצמו וג"כ כפול הא' על הב' וכפלם על ב' ותקבץ הכל הנה העולה יהיה שוה אל המספר המחולק כפול על עצמו | |
| Elements/Elements II-4 | reference | ספר_האלזיברא#Eeue | It was already clarified in [[ספר_היסודות_לאקלידס#Elements_II_4|'''Euclid, Elements, Book II, proposition 4''']] that: וכבר נתבאר ב'''תמונת הרביעית מן המאמר השני לאקלידס''' | |
| Elements/Elements II-4 | definition | ספר_האלזיברא#JPnC | When a straight line is cut randomly into two segments, the square on the whole line equals the sum of the two squares that are generated from the two segments plus twice the rectangle encompassed by the two segments.
: כי כאשר נחלק קו ישר לב' חלקים איך שקרה הנה מרבע הקו כלו שוה לשני המרבעים ההווים משני החלקים ולכפל השטח הנצב הזויות אשר יקיפו בו שני החלקים
כי כאשר נחלק קו ישר לב' חלקים איך שקרה הנה מרבע הקו כלו שוה לשני המרבעים ההווים משני החלקים ולכפל השטח הנצב הזויות אשר יקיפו בו שני החלקים | |
| Elements/Elements II-4 | (a+b)²=a²+b²+2(a·b) | לקוטים_מספר_פראלוקא#lAxq | If you have a number and you divide it into two unequal parts, I say that if you sum the [parts] that are multiplied by themselves, then you multiply the smaller [part] by the greater, multiply the product by 2 and sum it with the reserved, the result is equal to the [original] number multiplied by itself.
:![\scriptstyle\left(a+b\right)^2=a^2+b^2+\left[2\sdot\left(a\sdot b\right)\right]](/mediawiki/images/math/7/2/7/727b0c35fd93a7a347bed8413905049f.png) 169 אם יש לך מספר ותחלקהו על ב' חלקים בלתי שוים אומר כי אם תקבץ המספרי' המוכפלים על עצמם ואח"כ תכפול המספר הקטון על הגדול והעולה כפול על ב' ותקבץ זה עם השמור יעלה הכל כמו המספר כפול על עצמו
169 אם יש לך מספר ותחלקהו על ב' חלקים בלתי שוים אומר כי אם תקבץ המספרי' המוכפלים על עצמם ואח"כ תכפול המספר הקטון על הגדול והעולה כפול על ב' ותקבץ זה עם השמור יעלה הכל כמו המספר כפול על עצמו | |
| Elements/Elements II-4 | (a+b)²=a²+b²+2(a·b) | ספר_המלכים#wUeW | For any number divided into [two] parts, whichever they may be, the product of the whole number by itself is equal to the sum of the products of each of the two parts by itself and double the product of one of the two parts by the other.
:![\scriptstyle{\color{OliveGreen}{\left(a+b\right)^2=a^2+b^2+\left[2\sdot\left(a\sdot b\right)\right]}}](/mediawiki/images/math/d/f/2/df26d1cdaf50844081193e48666b1eeb.png) |style="width:45%; text-align:right;"|כל מספר יחלק בחלקים כמו שיהיו הנה הכאת המספר כלו בעצמו כמו הכאת כל אחד משני החלקים בעצמו וכפל הכאת אחד משני החלקים באחר כאשר יקובצו
|style="width:45%; text-align:right;"|כל מספר יחלק בחלקים כמו שיהיו הנה הכאת המספר כלו בעצמו כמו הכאת כל אחד משני החלקים בעצמו וכפל הכאת אחד משני החלקים באחר כאשר יקובצו | |
| Elements/Elements II-4 | 10,3+7 | ספר_המספר_/_אליהו_מזרחי#Ca24 | Example: the number ten, we divide it to three and seven. דמיון המספר עשרה חלקנוהו על שלשה ושבעה | |
| Elements/Elements II-4 | 12,4+8 | לקוטים_מספר_פראלוקא#HYCk | Example: we divide 12 to 4 and 8. המשל הנה נחלק 147vמספר י"ב על ד' ועל ח' | |
| Elements/Elements II-5 | 10,3+7 | ספר_החשבון_והמדות#zKhJ | Example: the number ten, we divide it to five and five, which are equal parts, then we divide it also to 7 and 3, which are unequal parts. דמיון המספר עשרה חלקנו לחמשה וחמשה שהם חלקים שוים גם חלקנוהו לז' וג' שהם חלקים בלתי שוים | |
| Elements/Elements II-5 | (½(a+b))²=(a·b)+(b-½(a+b))²=(a·b)+(½(a+b)-a)² | ספר_החשבון_והמדות#43lj | For any number divided into two equal parts and into two unequal parts, [the sum of] the product of one of the unequal parts by the other and the square of the difference between the two parts, i.e. between the equal part [= the half of the whole number] and the unequal [part] is equal to the square of half the [whole] number.
:![\scriptstyle{\color{OliveGreen}{\left(a\sdot b\right)+\left[\left[\frac{1}{2}\sdot\left(a+b\right)\right]-a\right]^2=\left(a\sdot b\right)+\left[b-\left[\frac{1}{2}\sdot\left(a+b\right)\right]\right]^2=\left[\frac{1}{2}\sdot\left(a+b\right)\right]^2}}](/mediawiki/images/math/7/0/c/70cf1effca1e2303b584ecf2bb737846.png) עוד כל מספר כאשר תחלקהו לשני חלקים שוים ולשני חלקים בלתי שוים הנה כפל החלק האחד אל חברו מהחלקים הבלתי שוים ומרובע מה שבין שני 32vהחלקים ר"ל בין החלק השוה ובלתי שוה שוה למרובע חצי המספר
עוד כל מספר כאשר תחלקהו לשני חלקים שוים ולשני חלקים בלתי שוים הנה כפל החלק האחד אל חברו מהחלקים הבלתי שוים ומרובע מה שבין שני 32vהחלקים ר"ל בין החלק השוה ובלתי שוה שוה למרובע חצי המספר | |
| Elements/Elements II-5 | (½(a+b))²=(a·b)+(b-½(a+b))²=(a·b)+(½(a+b)-a)² | ספר_מעשה_חושב#AHZr | The product of half the given number by itself is equal to [the sum of] the product of a part of that number by the other part and the square of the difference between one of the [unequal] parts and half of the [whole] given number. ח השטח ההוה מחצי המספר המונח בעצמו שוה לשטח ההוה מחלק מה מהמספר ההוא בחלק השני ולמרובע יתרון אחד מן החלקים על חצי המספר המונח | |
| Elements/Elements II-5 | (½(a+b))²=(a·b)+(b-½(a+b))²=(a·b)+(½(a+b)-a)² | ספר_המלכים#4PpZ | For any even number divided into halves and into [two] unequal parts, the product of half the [whole] number by itself is equal to [the sum of] the product of the greater part by the smaller [part] and the product of the excess of the half of the [whole] number over the smaller part by itself.
:![\scriptstyle{\color{OliveGreen}{\left[\frac{1}{2}\sdot\left(a+b\right)\right]^2=\left(a\sdot b\right)+\left[\left[\frac{1}{2}\sdot\left(a+b\right)\right]-a\right]^2}}](/mediawiki/images/math/d/b/e/dbe95ea8054f7a459368b72c0c2ba9fb.png) כל מספר זוג יחלק לחצאים ולחלקים מתחלפים הנה אשר יהיה מהכאת [חצי]M om. המספר בעצמו כמו ההווה מהכאת החלק הגדול בקטן עם הכאת מותר חצי המספר על החלק
כל מספר זוג יחלק לחצאים ולחלקים מתחלפים הנה אשר יהיה מהכאת [חצי]M om. המספר בעצמו כמו ההווה מהכאת החלק הגדול בקטן עם הכאת מותר חצי המספר על החלק | |
| Elements/Elements II-5 | (½(a+b))²=(a·b)+(b-½(a+b))²=(a·b)+(½(a+b)-a)² | לקוטים_מספר_פראלוקא#24rA | If you divide any number into two equal parts and into two unequal parts, the product of the equal parts one by the other is as the product of the unequal parts one by the other, when you add to it the [square] of the difference between the [equal] part and the [unequal part]. 160 אם תחלק מספר אחד לב' חלקים שוים ולב' חלקים בלתי שוים הנה כל כך יעלה כפל החלקים השוים זה על זה כמו כפל החלקים הבלתי שוים זה על זה ותוסיף בם כפל היתרון שיש מהחלק האחד על האחר | |
| Elements/Elements II-5 | 10,3+7 | ספר_המספר_/_אליהו_מזרחי#zYJb | Example: the number ten, we divide it to five and five, which are equal parts, then we divide it also to seven and three, which are unequal parts. דמיון המספר עשרה חלקנוהו לחמשה וחמשה שהם חלקים שוים גם חלקנוהו לשבעה ושלשה שהם חלקים בלתי שוים | |
| Elements/Elements II-5 | 10,3+7 | קצת_מענייני_חכמת_המספר#41Hj | Example: the number 10 that is divided into 7 and 3. במש' הי' שנחלק לז' ולג' | |
| Elements/Elements II-5 | reference | ספר_האלזיברא#7B0h | [[ספר_היסודות_לאקלידס#Elements_II_5|'''Euclid, Elements, Book II, proposition 5''']]: the square formed by AZ, which is four measures, and is as the square of half [the number of] the things that is known to be 16, is equal to surface BH, which is equal to the right-angled surface encompassed by the two unequal segments, whose area is known to be 12, plus the square formed by ZB that is the difference between the two parts.
:![\scriptstyle{\color{OliveGreen}{\left(a\sdot b\right)+\left[\left[\frac{1}{2}\sdot\left(a+b\right)\right]-b\right]^2=\left[\frac{1}{2}\sdot\left(a+b\right)\right]^2}}](/mediawiki/images/math/8/0/9/8094ef8a2f57faab6825944b3c1aef85.png) :
: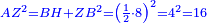 והנה כפי מה שנתבאר ב'''תמו' החמישית מן המאמר השני לאקלידס'''
והנה כפי מה שנתבאר ב'''תמו' החמישית מן המאמר השני לאקלידס''' | |
| Elements/Elements II-5 | (½(a+b))²=(a·b)+(b-½(a+b))²=(a·b)+(½(a+b)-a)² | קצת_מענייני_חכמת_המספר#9QNa | For any number divided into two equal parts and into two unequal parts, [the sum of] the product of one of the unequal parts by the other and the square of the difference between the two parts, i.e. between the equal part [= the half of the whole number] and the unequal [part] is equal to the square of half the [whole] number.
:![\scriptstyle{\color{OliveGreen}{\left(a\sdot b\right)+\left[\left[\frac{1}{2}\sdot\left(a+b\right)\right]-a\right]^2=\left[\frac{1}{2}\sdot\left(a+b\right)\right]^2}}](/mediawiki/images/math/9/3/3/9338487b12c6a0e6ee74fab15e061cb9.png) ד כל מספר כאשר תחלקהו לב' חלקים שוים ולב' חלקים בלתי שוים הנה הכאת החלק הא' עם חבירו מהחלקים הבלתי שוים ומרוב' מה שבין ב' חלקים ר"ל בין החלק השוה ובלתי שוה שוה למרובע חצי המספר
ד כל מספר כאשר תחלקהו לב' חלקים שוים ולב' חלקים בלתי שוים הנה הכאת החלק הא' עם חבירו מהחלקים הבלתי שוים ומרוב' מה שבין ב' חלקים ר"ל בין החלק השוה ובלתי שוה שוה למרובע חצי המספר | |
| Elements/Elements II-5 | 12,4+8 | לקוטים_מספר_פראלוקא#pZPT | Example: we divide 12 into two equal parts 6 and 6, and into two unequal parts 4 and 8. המשל נחלק מספר י"ב לב' חלקים שוים ו'ו' ולב' חלקים בלתי שוים ד' וח' | |
| Elements/Elements II-5 | (½(a+b))²=(a·b)+(b-½(a+b))²=(a·b)+(½(a+b)-a)² | ספר_המספר_/_אליהו_מזרחי#yKYx | For any number, when you divide it into two equal parts and into two unequal parts, [the sum of] the product of one of the unequal parts by the other and the square of the difference between the two parts, i.e. between the equal part [= the half of the whole number] and the unequal [part] is equal to the square of half the [whole] number.
:![\scriptstyle{\color{OliveGreen}{\left(a\sdot b\right)+\left[\left[\frac{1}{2}\sdot\left(a+b\right)\right]-a\right]^2=\left(a\sdot b\right)+\left[b-\left[\frac{1}{2}\sdot\left(a+b\right)\right]\right]^2=\left[\frac{1}{2}\sdot\left(a+b\right)\right]^2}}](/mediawiki/images/math/7/0/c/70cf1effca1e2303b584ecf2bb737846.png) עוד כל מספר כאשר תחלקהו לשני חלקים שוים ולשני חלקים בלתי שוים הנה הכאת החלק האחד עם חברו מהחלקים הבלתי שוים ומרובע מה שבין שני החלקים ר"ל בין החלק השוה ובלתי שוה שוה למרובע חצי המספר
עוד כל מספר כאשר תחלקהו לשני חלקים שוים ולשני חלקים בלתי שוים הנה הכאת החלק האחד עם חברו מהחלקים הבלתי שוים ומרובע מה שבין שני החלקים ר"ל בין החלק השוה ובלתי שוה שוה למרובע חצי המספר | |
| Elements/Elements II-6 | 12,4 | לקוטים_מספר_פראלוקא#qQuN | Example: we have 12, you divide it to 6 and 6, then add to 6 whichever number you wish. Suppose that we wish to add 4 to 6. המשל יש לנו מספר י"ב ותחלק אותו על ו"ו ותוסיף על ו' מספר איזה שתרצה ונניח כי נרצה להוסיף על ו' ד' | |
| Elements/Elements II-6 | reference | ספר_האלזיברא#BJrm | [[ספר_היסודות_לאקלידס#Elements_II_6|'''Euclid, Elements, Book II, proposition 6''']] that the right-angled surface encompassed by the whole line with the addition and the addition, which is equal to surface AW, whose area is known as 48, plus the square of half the line, whose area is known, which is 1, both together are 49, equals the square of the line formed by half the line with the addition, which is line AZ.
:![\scriptstyle{\color{OliveGreen}{\left[\left(a+b\right)\sdot b\right]+\left(\frac{1}{2}\sdot a\right)^2=\left[\left(\frac{1}{2}\sdot a\right)+b\right]^2}}](/mediawiki/images/math/a/9/2/a92046fe5abf282c91d09104586c53a7.png) וכבר נתבאר ב'''תמונה הששית 131vמן המאמר השני לאקלידס'''
וכבר נתבאר ב'''תמונה הששית 131vמן המאמר השני לאקלידס''' | |
| Elements/Elements II-6 | reference | ספר_האלזיברא#XaGL | [[ספר_היסודות_לאקלידס#Elements_II_6|'''Euclid, Elements, Book II, proposition 6''']] that the right-angled surface encompassed by the whole line with the addition and the addition, which is equal to surface ZD, whose area is 2 in our example, with the square formed by half the line, which is 16 in our example, both together are 36, equals the square of the line formed by half the line with the addition, which is line TB in our illustration.
:![\scriptstyle{\color{OliveGreen}{\left[\left(a+b\right)\sdot b\right]+\left(\frac{1}{2}\sdot a\right)^2=\left[\left(\frac{1}{2}\sdot a\right)+b\right]^2}}](/mediawiki/images/math/a/9/2/a92046fe5abf282c91d09104586c53a7.png) וכבר נתבאר ב'''תמונה הששית מן המאמר השני לאקלידס'''
וכבר נתבאר ב'''תמונה הששית מן המאמר השני לאקלידס''' | |
| Elements/Elements II-6 | 10,2 | ספר_החשבון_והמדות#yd9c | Example: the number ten, we divide it into two halves, which are five each, then we add two to the ten, they are 12. דמיון המספר עשרה וחלקנוהו לשני חצאים שהם כל חצי חמשה {{#annot:term|178,1206|GTDu}}הוספנו{{#annotend:GTDu}} על העשרה שנים והיו י"ב | |
| Elements/Elements II-6 | (a+b)·b+(½a)²=(½a+b)² | ספר_המלכים#c7yY | For any number divided into two halves and another number is added to it, the product of half the number and the additional [number] together by itself is equal to [the sum of] the product of the [whole] number plus the additional [number] by the additional [number] and the product of half the original number by itself.
:![\scriptstyle{\color{OliveGreen}{\left[\left(\frac{1}{2}\sdot a\right)+b\right]^2=\left[\left(a+b\right)\sdot b\right]+\left(\frac{1}{2}\sdot a\right)^2}}](/mediawiki/images/math/8/5/e/85ec1e0db93c2d4e5ffad82b081e922d.png) כל מספר זוג יחלק לשני חצאים ויתוסף בו מספר אחר הנה הכאת חצי המספר עם התוספת בכמהו כהכאת המספר עם התוספת בתוספת והכאת חצי המספר הראשון בעצמו
כל מספר זוג יחלק לשני חצאים ויתוסף בו מספר אחר הנה הכאת חצי המספר עם התוספת בכמהו כהכאת המספר עם התוספת בתוספת והכאת חצי המספר הראשון בעצמו | |
| Elements/Elements II-6 | 10,2 | ספר_המספר_/_אליהו_מזרחי#bDvj | Example: the number ten, we divide it into two halves, which are five each, then we add two to the ten, they are 12. דמיון המספר עשרה וחלקנוהו לשני חצאים שהם כל חצי חמשה הוספנו על העשרה שנים והיו י"ב | |
| Elements/Elements II-6 | (a+b)·b+(½a)²=(½a+b)² | לקוטים_מספר_פראלוקא#77le | If you divide any number into two equal parts, then add an additional [number] to the divided number, multiply [the sum] by the additional [number] and add [the product] to the square of one of the parts, I say that it is equal to the square of half [the number] with the additional [number].
:![\scriptstyle\left[\left(a+b\right)\sdot b\right]+\left(\frac{1}{2}\sdot a\right)^2=\left[\left(\frac{1}{2}\sdot a\right)+b\right]^2](/mediawiki/images/math/a/0/b/a0b403dd8b7c15a5cb1dc47a5f8ca5bb.png) 161 אם תחלק איזה מספר לב' חלקים שוים ותוסיף בם א' מהחלקי' כפול על עצמו ותוסיף בם התוספת על כל המספר המחולק ותכפול אותו המספר אשר הוספת ותחברם עם כפל אחד מהחלקים אומר כי הוא שוה אל כפל המחצית עם התוספת
161 אם תחלק איזה מספר לב' חלקים שוים ותוסיף בם א' מהחלקי' כפול על עצמו ותוסיף בם התוספת על כל המספר המחולק ותכפול אותו המספר אשר הוספת ותחברם עם כפל אחד מהחלקים אומר כי הוא שוה אל כפל המחצית עם התוספת | |
| Elements/Elements II-6 | (a+b)·b+(½a)²=(½a+b)² | קצת_מענייני_חכמת_המספר#nmaV | If we divide any number into half and add to it another number, [the sum of] the product of the whole number plus the additional [number] by the additional [number] and the square of half the number is equal to the square of half the number and the additional [number] together.
:![\scriptstyle{\color{OliveGreen}{\left[\left(a+b\right)\sdot b\right]+\left(\frac{1}{2}\sdot a\right)^2=\left[\left(\frac{1}{2}\sdot a\right)+b\right]^2}}](/mediawiki/images/math/a/9/2/a92046fe5abf282c91d09104586c53a7.png) ה כל מספר כאשר חלקנו אותו לחצי והוספת עליו מספר אחר הנה הכאת המספר כלו מחובר עם התוספ' בתוספת ומרובע חצי המספר שוה למרובע חצי המספר והתוספת ביחד
ה כל מספר כאשר חלקנו אותו לחצי והוספת עליו מספר אחר הנה הכאת המספר כלו מחובר עם התוספ' בתוספת ומרובע חצי המספר שוה למרובע חצי המספר והתוספת ביחד | |
| Elements/Elements II-6 | (a+b)·b+(½a)²=(½a+b)² | ספר_החשבון_והמדות#A9xw | If you divide any number into half and add to it another number, [the sum of] the product of the whole number plus the additional [number] by the additional [number] and the square of half the number is equal to the square of half the number and the additional [number] together.
:![\scriptstyle{\color{OliveGreen}{\left[\left(a+b\right)\sdot b\right]+\left(\frac{1}{2}\sdot a\right)^2=\left[\left(\frac{1}{2}\sdot a\right)+b\right]^2}}](/mediawiki/images/math/a/9/2/a92046fe5abf282c91d09104586c53a7.png) עוד כל מספר כאשר חלקת אותו לחצאים והוספת עליו מספר אחר הנה כפל המספר כלו מקובץ עם התוספת בתוספת והמרובע ההוה מחצי המספר שוה למרובע חצי המספר והתוספת ביחד
עוד כל מספר כאשר חלקת אותו לחצאים והוספת עליו מספר אחר הנה כפל המספר כלו מקובץ עם התוספת בתוספת והמרובע ההוה מחצי המספר שוה למרובע חצי המספר והתוספת ביחד | |
| Elements/Elements II-6 | (a+b)·b+(½a)²=(½a+b)² | ספר_מעשה_חושב#vsiV | When a number is divided into two halves and a number is added to it, [the sum of] the product of the additional [number] by the whole number plus the additional [number] and the square of half the number is equal to the square of half the number and the additional [number] summed together.
:![\scriptstyle\left[\left(a+b\right)\sdot b\right]+\left(\frac{1}{2}\sdot a\right)^2=\left[\left(\frac{1}{2}\sdot a\right)+b\right]^2](/mediawiki/images/math/a/0/b/a0b403dd8b7c15a5cb1dc47a5f8ca5bb.png) ה כאשר חולק מספר מה לחציין והוסף עליו מספר מה הנה שטח התוספת במספר כלו עם התוספת עם מרובע חצי המספר שוה למרובע חצי המספר והתוספת מקובצים
ה כאשר חולק מספר מה לחציין והוסף עליו מספר מה הנה שטח התוספת במספר כלו עם התוספת עם מרובע חצי המספר שוה למרובע חצי המספר והתוספת מקובצים | |
| Elements/Elements II-6 | (a+b)·b+(½a)²=(½a+b)² | ספר_המספר_/_אליהו_מזרחי#tcs6 | For any number, when you divide it into half and add to it another number, [the sum of] the product of the whole number plus the additional [number] by the additional [number] and the square of half the number is equal to the square of half the number and the additional [number] together.
:![\scriptstyle{\color{OliveGreen}{\left[\left(a+b\right)\sdot b\right]+\left(\frac{1}{2}\sdot a\right)^2=\left[\left(\frac{1}{2}\sdot a\right)+b\right]^2}}](/mediawiki/images/math/a/9/2/a92046fe5abf282c91d09104586c53a7.png) עוד כל מספר כאשר חלקת אותו לחצי והוספת עליו מספר אחר הנה הכאת המספר כלו מקובץ עם התוספת בתוספת והמרובע ההווה מחצי המספר שוה למרובע חצי המספר והתוספת ביחד
עוד כל מספר כאשר חלקת אותו לחצי והוספת עליו מספר אחר הנה הכאת המספר כלו מקובץ עם התוספת בתוספת והמרובע ההווה מחצי המספר שוה למרובע חצי המספר והתוספת ביחד | |
| Elements/Elements II-7 | (a+b)²+a²=(2·(a+b)·a)+b² | ספר_החשבון_והמדות#hdYZ | For any number divided into two parts, the sum of the square of the whole number and the square of one of the parts is equal to twice the product of this part by the whole number plus the product of the other part by itself.
:![\scriptstyle{\color{OliveGreen}{\left(a+b\right)^2+a^2=2\sdot\left[\left(a+b\right)\sdot a\right]+b^2}}](/mediawiki/images/math/0/3/a/03a8d96192812ea7db90d5166ad5177a.png) עוד כל מספר כשתחלקהו בשני חלקים איך שיקרה הנה המרובע ההוה מן המספר כלו והמרובע ההוה מאחד משני חלקים כאשר {{#annot:term|178,1216|tLsc}}התקבצו{{#annotend:tLsc}} שוים לכפל המספר כלו עם החלק הנזכר פעמים והמרובע ההוה מן החלק השני
עוד כל מספר כשתחלקהו בשני חלקים איך שיקרה הנה המרובע ההוה מן המספר כלו והמרובע ההוה מאחד משני חלקים כאשר {{#annot:term|178,1216|tLsc}}התקבצו{{#annotend:tLsc}} שוים לכפל המספר כלו עם החלק הנזכר פעמים והמרובע ההוה מן החלק השני | |
| Elements/Elements II-7 | 10,3+7 | ספר_החשבון_והמדות#L1Xh | Example: the number ten, we divide it randomly to seven and three. דמיון המספר עשרה וחלקנוהו איך שקרה על שבעה ועל שלשה | |
| Elements/Elements II-7 | (a+b)²+a²=(2·(a+b)·a)+b² | לקוטים_מספר_פראלוקא#lBZs | If you have a number and you divide it into two unequal parts, you multiply the greater part by itself and multiply also the divided number by itself, then sum both products, the result is equal to the product of the greater number by the divided number multiplied by two, then you add to it the square of smaller part.
:![\scriptstyle\left(a+b\right)^2+a^2=2\sdot\left[\left(a+b\right)\sdot a\right]+b^2](/mediawiki/images/math/5/e/f/5ef738b800e1b84fc257df70a83fb86b.png) 162 אם יש לך מספר ותחלקהו לב' חלקים בלתי שוים ותכפול החלק הגדול על עצמו גם תכפול המספר המחולק על עצמו ותחבר הב' הכפלות הנה העולה יהיה שוה אל כפל המספר הגדול על המספר המחולק ותכפלהו אח"כ על ב' גם תוסיף על זה כפל החלק הקטן ותחבר הכל יהיה שוה
162 אם יש לך מספר ותחלקהו לב' חלקים בלתי שוים ותכפול החלק הגדול על עצמו גם תכפול המספר המחולק על עצמו ותחבר הב' הכפלות הנה העולה יהיה שוה אל כפל המספר הגדול על המספר המחולק ותכפלהו אח"כ על ב' גם תוסיף על זה כפל החלק הקטן ותחבר הכל יהיה שוה | |
| Elements/Elements II-7 | (a+b)²+a²=(2·(a+b)·a)+b² | ספר_המספר_/_אליהו_מזרחי#WZG6 | For any number, when you divide it into two parts randomly, [the sum of] the square of the whole number and the square of one of the two parts is equal to twice the product of the whole number by the mentioned part plus the square of the second part.
:![\scriptstyle{\color{OliveGreen}{\left(a+b\right)^2+a^2=2\sdot\left[\left(a+b\right)\sdot a\right]+b^2}}](/mediawiki/images/math/0/3/a/03a8d96192812ea7db90d5166ad5177a.png) עוד כל מספר כאשר תחלקהו בשני חלקים איך שיקרה המרובע ההווה מן המספר כלו והמרובה ההווה מאחד משנים החלקים כאשר התקבצו שוים להכאת המספר כלו עם החלק הנזכר פעמים והמרובע ההווה מן החלק השני
עוד כל מספר כאשר תחלקהו בשני חלקים איך שיקרה המרובע ההווה מן המספר כלו והמרובה ההווה מאחד משנים החלקים כאשר התקבצו שוים להכאת המספר כלו עם החלק הנזכר פעמים והמרובע ההווה מן החלק השני | |
| Elements/Elements II-7 | 10,3+7 | קצת_מענייני_חכמת_המספר#nE0O | Example: the number 10 is divided into 7 and 3. במשל הרי הי' נחלק לז' ולג' | |
| Elements/Elements II-7 | 10,3+7 | ספר_המספר_/_אליהו_מזרחי#VZVn | Example: the number ten, we divide it randomly to 7 and 3. דמיון המספר עשרה וחלקנוהו איך שקרה על ז' ועל ג' | |
| Elements/Elements II-7 | 12,4+8 | לקוטים_מספר_פראלוקא#R7rS | Example: we wish to divide 12 to 4 and 8. המשל נרצה לחלק י"ב על ח' וד' | |
| Elements/Elements II-7 | (a+b)²+a²=(2·(a+b)·a)+b² | קצת_מענייני_חכמת_המספר#DEFA | For any number divided into two parts, the sum of the square of the whole number and the square of one of the parts is equal to twice the product of this part by the whole number plus the product of the other part by itself : ![\scriptstyle{\color{OliveGreen}{\left(a+b\right)^2+a^2=2\sdot\left[\left(a+b\right)\sdot a\right]+b^2}}](/mediawiki/images/math/0/3/a/03a8d96192812ea7db90d5166ad5177a.png) ו כל מספר שתחלקהו בב' חלקים איך שקרה המרובע ההווה מהמספר כלו והמרובע ההווה מא' מב' אלו החלקים כאשר התקבצו שוה להכאת החלק הנזכ' עם המספר כלו ולהכאת החלק הב' הנשאר בעצמו
ו כל מספר שתחלקהו בב' חלקים איך שקרה המרובע ההווה מהמספר כלו והמרובע ההווה מא' מב' אלו החלקים כאשר התקבצו שוה להכאת החלק הנזכ' עם המספר כלו ולהכאת החלק הב' הנשאר בעצמו | |
| Elements/Elements II-7 | definition | ספר_האלזיברא#cvaS | When a straight line is cut randomly into two segments, the sum of the squares on both segments equals twice the rectangle encompassed by both segments plus the square that is generated from the excess of the larger segment over the smaller segment. [ [[ספר_היסודות_לאקלידס#Elements_II_7|'''Euclid, Elements, Book II, proposition 7''']] ]
: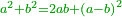 כי כאשר נחלק קו ישר 128rלשני חלקים איך שקרה הנה מרבעי שני החלקים שוים לכפל השטח הנצב הזויות אשר יקיפו בו שני החלקים ולמרבע ההוה ממותר החלק הגדול על הקטן
כי כאשר נחלק קו ישר 128rלשני חלקים איך שקרה הנה מרבעי שני החלקים שוים לכפל השטח הנצב הזויות אשר יקיפו בו שני החלקים ולמרבע ההוה ממותר החלק הגדול על הקטן | |
| Elements/Elements II-7 | (a+b)²+a²=(2·(a+b)·a)+b² | ספר_המלכים#isjp | For any number divided into two parts, [the sum of] the product of the [whole] number by itself and [the product of] one of the two parts by itself is equal to twice the product of the [whole] number by the part that is multiplied by itself plus the [product of the] other part by itself.
:![\scriptstyle{\color{OliveGreen}{\left(a+b\right)^2+a^2=\left[2\sdot\left(a+b\right)\sdot a\right]+b^2}}](/mediawiki/images/math/5/0/0/5002e3f78e2c682da17cd0da9b8a0cf2.png) כל מספר יחלק לשני חלקים
כל מספר יחלק לשני חלקים | |
| Elements/Elements II-8 | 10,3+7 | קצת_מענייני_חכמת_המספר#9UoH | Example: the number 10 is divided into 7 and 3. במשל הי' נחלק לז' ולג' | |
| Elements/Elements II-8 | 12,4+8 | לקוטים_מספר_פראלוקא#NtVp | Example: we wish to divide 12 to 4 and 8. המשל נרצה לחלק י"ב על ד' וח' | |
| Elements/Elements II-8 | 10,3+7 | ספר_המספר_/_אליהו_מזרחי#pqrO | Example: we have the number ten, we divide it randomly to 3 and 7. דמיון יש לנו מספר עשרה וחלקנוהו איך שהזדמן על ג' ועל ז' | |
| Elements/Elements II-8 | (4·(a+b)·a)+b²=((a+b)+a)² | ספר_המספר_/_אליהו_מזרחי#LHo4 | For any number, when you divide it into two parts randomly and you multiply the whole number by one of the two parts four times, then sum [the product] with the square of the other part [it] is equal to the [square] of the whole number plus the mentioned part when you sum them together.
:![\scriptstyle{\color{OliveGreen}{4\sdot\left[\left(a+b\right)\sdot a\right]+b^2=\left[\left(a+b\right)+a\right]^2}}](/mediawiki/images/math/c/a/f/caf2f89aa2a00ea5e45e6eed3d4bc694.png) עוד כל מספר כאשר חלקת אותו בשני חלקים איך שיקרה והכית המספר כלו עם אחד משני חלקיו ארבעה פעמים וחברת אותו עם מרובע החלק הנשאר שוה למרובע ההווה מן המספר כלו והחלק הנזכר כאשר תחברם ביחד
עוד כל מספר כאשר חלקת אותו בשני חלקים איך שיקרה והכית המספר כלו עם אחד משני חלקיו ארבעה פעמים וחברת אותו עם מרובע החלק הנשאר שוה למרובע ההווה מן המספר כלו והחלק הנזכר כאשר תחברם ביחד | |
| Elements/Elements II-8 | 10,3+7 | ספר_החשבון_והמדות#AJOP | Example: we have the number ten, we divide it randomly to 3 and 7. דמיון יש לנו מספר מנינו העשרה וחלקנוהו איך שהזדמן על ג' ועל ז' | |
| Elements/Elements II-8 | (4·(a+b)·a)+b²=((a+b)+a)² | ספר_המלכים#Ocvs | For any number divided into two parts and one of the two parts is added to it, the product of the [whole] number plus the additional [part] by itself is equal to [the sum of] the product of the [whole] number by the additional [part] four times and the product of the other part by itself.
:![\scriptstyle{\color{OliveGreen}{\left[\left(a+b\right)+a\right]^2=\left[4\sdot\left(a+b\right)\sdot a\right]+b^2}}](/mediawiki/images/math/3/4/8/348f212d9f2bb23aebbed2132e172f55.png) כל מספר יחלק בשני חלקים ונוסף עליו כמו אחד משני החלקים הנה הכאת המספר עם התוספת בכמהו כהכאת המספר בתוספת ד' פעמים והכאת החלק האחר בכמהו
כל מספר יחלק בשני חלקים ונוסף עליו כמו אחד משני החלקים הנה הכאת המספר עם התוספת בכמהו כהכאת המספר בתוספת ד' פעמים והכאת החלק האחר בכמהו | |
| Elements/Elements II-8 | (4·(a+b)·a)+b²=((a+b)+a)² | לקוטים_מספר_פראלוקא#TRuX | If you have a number and you divide it into unequal two parts, if you add one of the parts to the whole number, i.e. the divided number, [its square] is equal to the same part that you added multiplied by the whole number, then multiplied by four, when you add to it the square of the second part.
:![\scriptstyle\left[\left(a+b\right)+a\right]^2=4\sdot\left[\left(a+b\right)\sdot a\right]+b^2](/mediawiki/images/math/b/3/2/b32320b109ede228563dccc608b448d3.png) 163 אם יש לך מספר ותחלקהו לב' חלקים בלתי שוים אם תוסיף א' מהחלקים על כל המספר ר"ל על המספר המחולק יהיה שוה אל אותו החלק אשר הוספת כפול על כל המספר אח"כ העולה תכפול על ד' ותוסיף על זה מרובע החלק השני
163 אם יש לך מספר ותחלקהו לב' חלקים בלתי שוים אם תוסיף א' מהחלקים על כל המספר ר"ל על המספר המחולק יהיה שוה אל אותו החלק אשר הוספת כפול על כל המספר אח"כ העולה תכפול על ד' ותוסיף על זה מרובע החלק השני | |
| Elements/Elements II-8 | (4·(a+b)·a)+b²=((a+b)+a)² | קצת_מענייני_חכמת_המספר#R68t | For any number divided into two parts as you wish, if you multiply the whole number by one of the parts four times, the sum of the product with the square of the other part is equal to the [square] of the whole number plus the one part
:![\scriptstyle{\color{OliveGreen}{4\sdot\left[\left(a+b\right)\sdot a\right]+b^2=\left[\left(a+b\right)+a\right]^2}}](/mediawiki/images/math/c/a/f/caf2f89aa2a00ea5e45e6eed3d4bc694.png) ז כל מספר שחלקת אותו לב' חלקים איך שקרה אם הכית המספר כלו עם חלק אח' מהם ד' פעמים וקבצת הכל עם מרובע החלק הב' הנשאר היה שוה להכאת מספרו החלק הנזכר כאשר תחברם יחד
ז כל מספר שחלקת אותו לב' חלקים איך שקרה אם הכית המספר כלו עם חלק אח' מהם ד' פעמים וקבצת הכל עם מרובע החלק הב' הנשאר היה שוה להכאת מספרו החלק הנזכר כאשר תחברם יחד | |
| Elements/Elements II-8 | (4·(a+b)·a)+b²=((a+b)+a)² | ספר_החשבון_והמדות#EAD6 | For any number divided into two parts as you wish, if you multiply the whole number by one of the parts four times, the sum of the product with the square of the other part is equal to the [square] of the whole number plus the one part.
:![\scriptstyle{\color{OliveGreen}{4\sdot\left[\left(a+b\right)\sdot a\right]+b^2=\left[\left(a+b\right)+a\right]^2}}](/mediawiki/images/math/c/a/f/caf2f89aa2a00ea5e45e6eed3d4bc694.png) עוד כל מספר כאשר חלקת אותו בשני חלקים איך שיקרה וכפלת המספר כלו עם אחד משני חלקיו ארבעה פעמים ועם מרובע החלק הנשאר שוה למרובע ההוה מן המספר כלו והחלק הנזכר כאשר תחברם ביחד ותקח מרובעם
עוד כל מספר כאשר חלקת אותו בשני חלקים איך שיקרה וכפלת המספר כלו עם אחד משני חלקיו ארבעה פעמים ועם מרובע החלק הנשאר שוה למרובע ההוה מן המספר כלו והחלק הנזכר כאשר תחברם ביחד ותקח מרובעם | |
| Elements/Elements II-9 | 10,3+7 | ספר_המספר_/_אליהו_מזרחי#LnLo | Example: the number 10, we divide it into two equal parts, which are 5, and into two unequal parts, which are 7 and 3. דמיון המספר י' וחלקנוהו לשני חלקים שוים והם ה' ולשני חלקים בלתי שוים והם ז' ג' | |
| Elements/Elements II-9 | 12,4+8 | לקוטים_מספר_פראלוקא#QBwh | Example: we wish to divide 12 into two equal parts 6 and 6 and into two unequal parts 8 and 4. המשל נרצה לחלק מספר י"ב לב' חלקי' שוים ו'ו' ולב' חלקים בלתי שוים ח' ד' | |
| Elements/Elements II-9 | a²+b²=2·((½·(a+b))²+(a-(½·(a+b)))²) | לקוטים_מספר_פראלוקא#E95L | If you divide any line into two equal parts and into two unequal parts, then you multiply each of the unequal parts [by itself] and sum them, they are twice [the sum of] the product of the equal parts plus the [square of] excess of the greater [part] over [half the number].
:![\scriptstyle a^2+b^2=2\sdot\left[\left[\frac{1}{2}\sdot\left(a+b\right)\right]^2+\left[a-\left[\frac{1}{2}\sdot\left(a+b\right)\right]\right]^2\right]](/mediawiki/images/math/5/e/8/5e87deb6f6b5cec598e43b4b3374a25c.png) 164 אם תחלק איזה קו לב' חלקים שוים ולב' חלקים בלתי שוים וכפול כל אחד מהחלקים בלתי שוים ותחברם הם כפל מהחלקים השוים נוסף בם יתרון החלק הגדול על הקטן
164 אם תחלק איזה קו לב' חלקים שוים ולב' חלקים בלתי שוים וכפול כל אחד מהחלקים בלתי שוים ותחברם הם כפל מהחלקים השוים נוסף בם יתרון החלק הגדול על הקטן | |
| Elements/Elements II-9 | a²+b²=2·((½·(a+b))²+(a-(½·(a+b)))²) | ספר_המספר_/_אליהו_מזרחי#ClRv | For any number that you divide into two equal parts and into two unequal parts, [the sum of] the two squares of the unequal parts is equal to twice [the sum of] the square of half the [whole] number and [the square] of the excess of the large part over the half [of the whole number].
:![\scriptstyle{\color{OliveGreen}{a^2+b^2=2\sdot\left[\left[\frac{1}{2}\sdot\left(a+b\right)\right]^2+\left[a-\left[\frac{1}{2}\sdot\left(a+b\right)\right]\right]^2\right]}}](/mediawiki/images/math/3/7/c/37cd2d64c6505f872e3604129685d3bc.png) עוד כל מספר שחלקת אותו לשני חלקים שוים ושני חלקים בלתי שוים הנה שני המרובעים אשר יהיו מהחלקים הבלתי שוים הם כפל שני המרובעים אשר יהיו מחצי המספר ומהתוספת אשר לחלק הגדול על הה' שהוא המחצית
עוד כל מספר שחלקת אותו לשני חלקים שוים ושני חלקים בלתי שוים הנה שני המרובעים אשר יהיו מהחלקים הבלתי שוים הם כפל שני המרובעים אשר יהיו מחצי המספר ומהתוספת אשר לחלק הגדול על הה' שהוא המחצית | |
| Elements/Elements II-9 | a²+b²=2·((½·(a+b))²+(a-(½·(a+b)))²) | ספר_החשבון_והמדות#CM8j | For any number divided into two equal parts and into two unequal parts, [the sum of] the squares of the unequal parts is equal to twice [the sum of] the square of half the [whole] number and the square of the difference between the large part and the half [of the whole number].
:![\scriptstyle{\color{OliveGreen}{a^2+b^2=2\sdot\left[\left[\frac{1}{2}\sdot\left(a+b\right)\right]^2+\left[a-\left[\frac{1}{2}\sdot\left(a+b\right)\right]\right]^2\right]}}](/mediawiki/images/math/3/7/c/37cd2d64c6505f872e3604129685d3bc.png) עוד כל מספר שחלקת אותו לשני חלקים שוים ושני חלקים בלתי שוים הנה שני המרובעים אשר יהיו מהחלקים הבלתי שוים הם כפל שני המרובעים אשר יהיו מחצי המספר ומהתוספת אשר לחלק הגדול על המחצית
עוד כל מספר שחלקת אותו לשני חלקים שוים ושני חלקים בלתי שוים הנה שני המרובעים אשר יהיו מהחלקים הבלתי שוים הם כפל שני המרובעים אשר יהיו מחצי המספר ומהתוספת אשר לחלק הגדול על המחצית | |
| Elements/Elements II-9 | 10,3+7 | ספר_החשבון_והמדות#O8o8 | Example: we have the number ten, we divide it into two equal parts, which are 5, and into two unequal parts, which are 3 and 7. דמיון יש לנו מספר מנינו עשרה וחלקנוהו לשני חלקים שוים על ה' ושני חלקים בלתי שוים על ג' וז' | |
| Elements/Elements II-9 | a²+b²=2·((½·(a+b))²+(a-(½·(a+b)))²) | ספר_המלכים#g6A2 | For any even number divided into two halves and into two unequal parts, [the sum of the products of] each of the two unequal parts by themselves is equal to [the sum of] twice the product of half the [whole] number by itself and twice the product of the excess of half the [whole] number over the smaller part by itself.
:![\scriptstyle{\color{OliveGreen}{a^2+b^2=\left[2\sdot\left[\frac{1}{2}\sdot\left(a+b\right)\right]^2\right]+\left[2\sdot\left[\left[\frac{1}{2}\sdot\left(a+b\right)\right]-a\right]^2\right]}}](/mediawiki/images/math/2/a/b/2abb4b772df68504faed6649a992d8d3.png) כל מספר זוג יחלק בשני חצאים ובשני חלקים מתחלפים הנה כל אחד משני החלקים המתחלפים בכמהו כהכאת חצי המספר בכמהו שני פעמים והכאת מותר חצי המספר על החלק הקטן בכמהו שני פעמים
כל מספר זוג יחלק בשני חצאים ובשני חלקים מתחלפים הנה כל אחד משני החלקים המתחלפים בכמהו כהכאת חצי המספר בכמהו שני פעמים והכאת מותר חצי המספר על החלק הקטן בכמהו שני פעמים | |
| Elements/Elements-Introduction | a=b,a-c=b-c | ספר_המספר_/_אליהו_מזרחי#tNTC | For, when equal is subtracted from equals, then the remainders are necessarily equal, according to what is clarified in the introduction of the first section of Euclid's [book].
: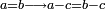 כי כאשר יחוסר מהשוים שוה יהיו הנשארים שוים בהכרח לפי מה שהתבאר בפתיחת המאמר הראשון מאקלידס
כי כאשר יחוסר מהשוים שוה יהיו הנשארים שוים בהכרח לפי מה שהתבאר בפתיחת המאמר הראשון מאקלידס | |
| Elements/Elements-Introduction | a=b,a-c=b-c | ספר_המספר_/_אליהו_מזרחי#gaDw | This is because it was already clarified in the introduction of Euclid's book that when equal is subtracted from equals, then the remainders are equal
: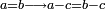 וזה שכבר התבאר בפתיחת ספר אקלידס כאשר חוסר מהשוים שוה יהיה הנשאר שוה
וזה שכבר התבאר בפתיחת ספר אקלידס כאשר חוסר מהשוים שוה יהיה הנשאר שוה | |
| Elements/Elements IX-21 | definition | ספר_המספר_/_אליהו_מזרחי#qiN5 | When even numbers are summed, as many as they may be, their sum is an even number. הוא כאשר נקבצו מספרי זוגות כמה שיהיו הנה קבוצם מספר זוג | |
| Elements/Elements IX-22 | definition | ספר_המספר_/_אליהו_מזרחי#yrIb | When odd numbers are summed, as many as they may be, and their multitude is even, their sum is an even number. וכאשר נקבצו מספרים נפרדים כמה שיהיו והיה מספרם זוג הנה קבוצם מספר זוג | |
| Elements/Elements IX-23 | definition | ספר_המספר_/_אליהו_מזרחי#1A6m | When odd numbers are summed, as many as they may be, and their multitude is odd, their sum is an odd number. וכאשר נקבצו מספרים נפרדים כמה שיהיו והיה מספרם מפרד הנה קבוצם נפרד | |
| Elements/Elements IX-28 | definition | ספר_המספר_/_אליהו_מזרחי#RVMm | When an even number is multiplied by an odd number, or by an even number, then the product is even.
: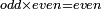 ; ;  הוא שכאשר הוכה מספר זוג במספר נפרד או במספר זוג הנה המקובץ זוג
הוא שכאשר הוכה מספר זוג במספר נפרד או במספר זוג הנה המקובץ זוג | |
| Elements/Elements IX-29 | definition | ספר_המספר_/_אליהו_מזרחי#DYPK | When an odd number is multiplied by an odd number, then the product is odd.
: וכאשר הוכה מספר נפרד במספר נפרד הנה המקובץ נפרד
וכאשר הוכה מספר נפרד במספר נפרד הנה המקובץ נפרד | |
| Elements/Elements V-15 | (n·a)÷(n·b)=a÷b | ספר_המספר_/_אליהו_מזרחי#PIBA | For, it was already clarified in Euclid's Book of Elements, according to what was preceded in this section, that the given numbers have the same ratio as the ratio of their equimultiples.
: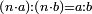 וזה שכבר התבאר בספר היסודות לאקלידס לפי מה שקדם מן המאמר שמהמספרים המונחים הם יחס קצתם אל קצת הוא כיחס כפליהם קצתם אל קצת
וזה שכבר התבאר בספר היסודות לאקלידס לפי מה שקדם מן המאמר שמהמספרים המונחים הם יחס קצתם אל קצת הוא כיחס כפליהם קצתם אל קצת | |
| Elements/Elements V-15 | (n·a)÷(n·b)=a÷b | ספר_המספר_/_אליהו_מזרחי#wUJB | For, it was already clarified in Euclid's Book of Elements, in the fifth section, that any numbers, whose multiples are equal, have the same ratio as the ratio of their equimultiples.
: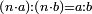 וזה שכבר התבאר בספר היסודות לאקלידס במאמר החמישי ממנו שהמספרים אשר כפליהם שוים הנה יחס קצתם אל קצת כיחס כפליהם קצתם אל קצת
וזה שכבר התבאר בספר היסודות לאקלידס במאמר החמישי ממנו שהמספרים אשר כפליהם שוים הנה יחס קצתם אל קצת כיחס כפליהם קצתם אל קצת | |
| Elements/Elements V-9 | definition | ספר_המספר_/_אליהו_מזרחי#2X92 | For, any two magnitudes, which have the same ratio to the same magnitude, necessarily equal one another, according to what is clarified in Euclid's Book of Elements.
: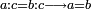 כי כל שני שעורים שיחסם אל שעור אחר בעצמו יחס אחד הנה הם שוים בהכרח לפי מה שהתבאר בספר היסודות לאקלידס
כי כל שני שעורים שיחסם אל שעור אחר בעצמו יחס אחד הנה הם שוים בהכרח לפי מה שהתבאר בספר היסודות לאקלידס | |
| Elements/Elements VI-17 | reference | ספר_האלזיברא#cH1a | [[ספר_היסודות_לאקלידס#Elements_VI_17|'''Euclid, Elements, Book VI, proposition 17''']] that the product of the first by the last is as the product of the mean by its similar.
: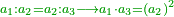 וכבר נתבאר מ'''תמונת י"ז מן המאמר הששי לאקלידס'''
וכבר נתבאר מ'''תמונת י"ז מן המאמר הששי לאקלידס''' | |
| Elements/Elements VI-17 | definition | ספר_האלזיברא#KlWg | It is already explained in {{#annot: reference | #Elements VI-17 | cH1a}}[[ספר_היסודות_לאקלידס#Elements_VI_17|'''Euclid, Elements, Book VI, proposition 17''']] that the product of the first by the last is as the product of the mean by its similar.
: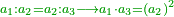 וכבר נתבאר מ'''תמונת י"ז מן המאמר הששי לאקלידס'''{{#annotend:cH1a}} כי {{#annot: term | #multiplication, #הכאה | poG8}}הכאת ה{{#annotend:poG8}}ראשו' באחרון כמו הכאת האמצעי בדומה לו
וכבר נתבאר מ'''תמונת י"ז מן המאמר הששי לאקלידס'''{{#annotend:cH1a}} כי {{#annot: term | #multiplication, #הכאה | poG8}}הכאת ה{{#annotend:poG8}}ראשו' באחרון כמו הכאת האמצעי בדומה לו | |
| Elements/Elements VII-11 | a÷b=c÷d→(c-a)÷(d-b) | ספר_המספר_/_אליהו_מזרחי#Iu2h | when two numbers are subtracted from two numbers and the ratio of the subtrahend to the subtrahend is the same as the ratio of the minuend to the minuend, then the [ratio of] the remainder to the remainder is the same as the ratio of the minuend to the minuend.
:: וזה שכבר התבאר בספר היסודות לאקלידס במאמר השביעי ממנו בתמונת י"א שכאשר חוסרו משני מספרים ב' מספרים והיה יחס המחוסר אל המחוסר כיחס הכל אל הכל הנה יהיה הנשאר אל הנשאר כיחס הכל אל הכל
וזה שכבר התבאר בספר היסודות לאקלידס במאמר השביעי ממנו בתמונת י"א שכאשר חוסרו משני מספרים ב' מספרים והיה יחס המחוסר אל המחוסר כיחס הכל אל הכל הנה יהיה הנשאר אל הנשאר כיחס הכל אל הכל | |
| Elements/Elements VIII-11 | definition | ספר_האלזיברא#QcHW | the ratio of a square to a square is as the ratio of its side to its side duplicated. [ [[ספר_היסודות_לאקלידס#Elements_VIII_11|'''Euclid, Elements, Book VIII, proposition 11''']] ]
: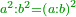 וזה כי מפני כי יחס מרבע אל מרבע כיחס צלעו אל צלעו שנוי
וזה כי מפני כי יחס מרבע אל מרבע כיחס צלעו אל צלעו שנוי | |
| Elements/Elements VIII-11 | definition | ספר_האלזיברא#Nl0O | This is because the ratio of a square to a square is the same as the ratio of its side to its side duplicate.
: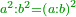 וזה מפני כי {{#annot: term | #ratio, #יחס | Eve2}}יחס{{#annotend:Eve2}} מרובע אל מרובע כיחס צלעו אל צלעו {{#annot: term | #duplicate, #שנוי | Q7dD}}שנוי{{#annotend:Q7dD}} ר"ל {{#annot: term | #multiplied, #כפול | G4iq}}כפול{{#annotend:G4iq}}
וזה מפני כי {{#annot: term | #ratio, #יחס | Eve2}}יחס{{#annotend:Eve2}} מרובע אל מרובע כיחס צלעו אל צלעו {{#annot: term | #duplicate, #שנוי | Q7dD}}שנוי{{#annotend:Q7dD}} ר"ל {{#annot: term | #multiplied, #כפול | G4iq}}כפול{{#annotend:G4iq}} | |
| Elements/Elements VIII-11 | reference | ספר_האלזיברא#D3f5 | [[ספר_היסודות_לאקלידס#Elements_VIII_11|'''Euclid, Elements, Book VIII, proposition 11''']] מתמונת י"א מן המאמר השמיני לאקלידס | |
| Elements/Elements VIII-11 | definition | ספר_המספר_/_אליהו_מזרחי#sPcq | Its reason is also known from what was clarified in Euclid's Book of Elements, in the eighth section that for every two squares numbers the ratio of one of them to the other is as the duplicate ratio of that which the side has to the side.
: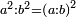 הנה סבתו ג"כ ידועה ממה שהתבאר בספר היסודו' לאקלידס במאמר הח' ממנו שכל שני מספרים מרובעים הנה יחס הא' מהם אל חברו הוא כיחס צלעו אל צלעו שנוי בכפל
הנה סבתו ג"כ ידועה ממה שהתבאר בספר היסודו' לאקלידס במאמר הח' ממנו שכל שני מספרים מרובעים הנה יחס הא' מהם אל חברו הוא כיחס צלעו אל צלעו שנוי בכפל | |
| Elements/Elements VIII-12 | reference | ספר_האלזיברא#jD6N | According to [[ספר_היסודות_לאקלידס#Elements_VIII_12|'''Euclid, Elements, Book VIII, proposition 12''']] מתמונת י"ב מן המאמר השמיני לאקלידס | |
| Elements/Elements VIII-12 | definition | ספר_האלזיברא#eDWE | This is because the ratio of a cube to a cube is the same as the ratio of its side to its side triplicate
: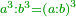 וזה מפני כי יחס מעקב אל מעקב כיחס צלעו אל צלעו {{#annot: term | #triplicate, #משולש | A27z}}משלש{{#annotend:A27z}}
וזה מפני כי יחס מעקב אל מעקב כיחס צלעו אל צלעו {{#annot: term | #triplicate, #משולש | A27z}}משלש{{#annotend:A27z}} | |
| Elements/Elements XIII-1 | definition | המחומשים_והמעושרים#1Da2 | Euclid proved that when a line is divided according to the ratio of a mean and two extremes, so that half the whole line is added to the larger segment, and all this is multiplied by itself, then the resulting square is equal to five times the square of half the line [Elements XIII.1]. ובאר אקלידס כי כאשר נחלק קו על יחס אמצעי ושתי קצוות שהחלק הגדול כאשר נוסף בארכו כמו חצי הקו כלו והוכה כל זה בעצמו המרובע ההוה מזה יהיה חמשה דמיוני המרובע ההוה מחצי הדבר | |
| Elements/Elements XIII-10 | definition | המחומשים_והמעושרים#w084 | We do this since Euclid has explained that the line that cuts the fifth is on the line that cuts the sixth and the tithe, when they are set on one circle [Elements XIII,10]. ועשינו זה בעבור כי באר אוקלידס כי קו החותך החמישית על הקו החותך הששית והעשירית כאשר הונחו בעגולה אחת | |
| Elements/Elements XIII-3 | definition | המחומשים_והמעושרים#mhLN | Euclid has already explained [Elements XIII.3] that when line BH is divided by the ratio of a mean and two extremes, so that the greater part is equal to line DH, it is known that when a line is divided by the ratio of a mean and two extremes and we add half the greater part to the smaller part, then multiply the whole sum by itself, the square formed by the sum of the two is five times the product of [half] the greater part by itself.
: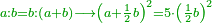 ובאר אוקלידס שקו ב"ה כאשר חולק על יחס אמצעי ושני קצוות שהחלק הגדול הוא שוה אל קו ד"ה והוא ידוע כי כאשר נחלק קו אחד על יחס בעל אמצעי ושני קצוות ונוסיף על החלק הקטן חצי החלק הגדול ונכה הכל בעצמו שהמרובע ההוה משני אלו מקובצים יהיה חמשה דמיוני הכאת החלק הגדול בעצמו
ובאר אוקלידס שקו ב"ה כאשר חולק על יחס אמצעי ושני קצוות שהחלק הגדול הוא שוה אל קו ד"ה והוא ידוע כי כאשר נחלק קו אחד על יחס בעל אמצעי ושני קצוות ונוסיף על החלק הקטן חצי החלק הגדול ונכה הכל בעצמו שהמרובע ההוה משני אלו מקובצים יהיה חמשה דמיוני הכאת החלק הגדול בעצמו | |
| Elements/Elements XIII-5 | definition | המחומשים_והמעושרים#3lPz | It is known from what we have said, as Euclid said, that for every line divisible according to the ratio of a mean and two extremes, when another part equal to the larger portion is added to the line, then whole [line] is divisible according to the ratio of a mean and two extremes, and the larger portion of which is the original line [Elements XIII.5]. והוא ידוע מאשר אמרנו שאמרו אקלידס ואמר שכל קו שהוא על יחס אמצעי ושני קצוות ונוסף באורך הקו כמו החלק 60rהגדול וכל זה יחלק על יחס אמצעי ושני קצוות שהחלק הגדול הוא הקו הראשון | |
| Elements/Elements XIII-9 | definition | המחומשים_והמעושרים#ScqD | It he said in another place that when the side of a hexagon and the side of a decagon inscribed in the same circle are united in one line, then this whole line is divisible according to the ratio of a mean and two extremes, and the side of the hexagon is the larger portion [Elements XIII.9]. ואמר במקום אחר שכאשר קובצו צלע המשושה עם צלע המעושר אשר בעגולה אחת בקו אחד ישר יהיה כל הקו נחלק על יחס אמצעי ושני קצוות וצלע המשושה הוא החלק הגדול | |
| solid/ellipsoid | ב.י.צ./ביצני | definition | ספר_יסודי_התבונה_ומגדל_האמונה#eg9d | that which is surrounded by a curved surface is called ellipsoid [lit. elliptic solid] or a solid that is similar to an ellipse [lit. egg-like solid]. ואשר יקיף אותו פרוש אחד עקום נקרא גולם ביצני או גולם דומה לביצה |
| solid/ellipsoid | ב.י.צ./ביצני | term | ספר_יסודי_התבונה_ומגדל_האמונה#gics | הגולם הביצני |
| solid/ellipsoid | ב.י.צ./דומה לביצה | term | ספר_יסודי_התבונה_ומגדל_האמונה#Rccp | הגולם הדומה לביצה |
| to encompass/encompassed | נ.ק.פ./הקיף | term | ספר_האלזיברא#noZK | אשר יקיפו בו קוי |
| to encompass/encompassed | נ.ק.פ./הקיף | term | ספר_האלזיברא#hrMO | אשר יקיפו בו שני החלקים |
| to encompass/encompassed | ר.ש.מ./נרשם | definition | ספר_היסודות_לאקלידס#9RjX | The figure is said to be circumscribed about a figure when each of its sides touches each of the respective angles of the figure about which it is circumscribed. ויאמר כי התמונה נרשמת סביב התמונה כשתהיה כל אחת מצלעותיה ממששת לכל אחת מזויות התמונה אשר היא נרשמת סביבה |
| geometry/end | כ.ל.י./תכלית | term | מלאכת_המספר#tYDo | תכליותיו |
| geometry/end | קצה | term | מלאכת_המספר#Idv3 | קצה |
| geometry/end | קצה | term | מלאכת_המספר#Ltil | קצה |
| geometry/end | ש.פ.י./שפה | term | מלאכת_המספר#pd7L | שפת |
| triangle/equilateral triangle | ש.ו.ה./שוה זויות | term | מלאכת_המספר#dBGu | משולש השוה הזויות |
| triangle/equilateral triangle | ש.ו.ה./שוה זויות | term | מלאכת_המספר#0szp | המשולש השוה הזויות |
| triangle/equilateral triangle | ש.ו.ה./שוה צלעות | term | ספר_הכללים_במספר#sp6o | משולש שוה הצלעות |
| triangle/equilateral triangle | ש.ו.ה./שוה צלעות | term | ספר_הכללים_במספר#Okmu | המשולש השוה הצלעות |
| line/extension | מ.ש.כ./המשכות | term | האריתמטיקה_של_ניקומכוס#I0sK | המשכותו |
| solid/face | צ.ד.ד./צד | term | ספר_יסודי_התבונה_ומגדל_האמונה#S4QN | צדים |
| solid/face | צ.ד.ד./צד | term | ספר_יסודי_התבונה_ומגדל_האמונה#TPlF | צדדין |
| solid/face | י.ש.ב./מושב | term | ספר_יסודי_התבונה_ומגדל_האמונה#H8Af | מושבותיהן |
| solid/face | י.ש.ב./מושב | term | ספר_יסודי_התבונה_ומגדל_האמונה#FTa7 | מושבות |
| solid/face | צ.ד.ד./צד | term | ספר_יסודי_התבונה_ומגדל_האמונה#OEA8 | צדין |
| surface/flat surface | י.ש.ר./ישר | definition | ספר_יסודי_התבונה_ומגדל_האמונה#WnsW | the plane surface is the drawn on the straightness of the lines that surround it and in parallel to them והשטח הישר הוא הנמשך על יושר הקוים המקיפים אותו ועל נכחם |
| surface/flat surface | י.ש.ר./משור | term | מלאכת_המספר#wOY6 | מישור |
| surface/flat surface | ש.ו.ה./שוה | definition | ספר_היסודות_לאקלידס#kmWD | The '''plane surface''' is that which lies straightly by the arrangement of straight lines on it one by one. והפשוט השוה הוא המוצב על נכוחות אי זה קוים ישרים יהיו עליו קצתם אל קצתם |
| geometry/geometrical shape | צ.ו.ר./צורה | term | קצור_המספר#Tj2a | צורתו |
| geometry/geometrical shape | תמונה | term | ספר_האלזיברא#sSIx | תמונה |
| geometry/geometrical shape | term | ספר_המספר_/_אברהם_אבן_עזרא#kCK2 | דמות | |
| geometry/geometrical shape | תמונה | term | חשבון_השטחים#DM4q | תמונת גימטריאות |
| geometry/geometrical shape | תמונה | term | תחבולות_המספר#QKAs | תמונה |
| geometry/geometrical shape | צ.ו.ר./צורה | term | ספר_ג'יבלי_אלמוקבאלא#NU0p | צורת ה |
| geometry/geometrical shape | תמונה | term | חשבון_השטחים#ld89 | תמונות גימאטריות |
| geometry/geometrical shape | צ.ו.ר./צורה | term | קצור_המספר#uLTa | צורת ה |
| geometry/geometrical shape | ש.ע.ר./שעורי | term | האנציקלופדיה_של_אבו_אלצלת#1yEx | התמונות השעוריות |
| geometry/geometrical shape | תמונה | definition | ספר_היסודות_לאקלידס#zvgX | The '''figure''' is that which is contained by a boundary or boundaries. והתמונה היא אשר יקיף אותה גבול או גבולים |
| geometry/geometrical shape | תמונה | term | תחבולות_המספר#nDez | תמונ' גימטרית |
| geometry/geometrical shape | תמונה | term | חשבון_השטחים#S3AT | תמונה |
| geometry/geometrical shape | תמונה | term | תחבולות_המספר#zsC7 | תמונות גימטריות |
| geometry/geometrical shape | תמונה | term | ספר_המספר_/_אליהו_מזרחי#A0eJ | תמונות |
| geometry/geometrical shape | תמונה | term | תחבולות_המספר#cUJQ | תמונות גימטריאות |
| geometry/geometrical shape | term | ספר_המספר_/_אברהם_אבן_עזרא#6zsx | צורות | |
| geometry/geometrical shape | צ.ו.ר./צורה | term | ספר_ג'יבלי_אלמוקבאלא#aHl6 | בצורה |
| geometry/geometrical shape | צ.ו.ר./צורה | term | ספר_ג'יבלי_אלמוקבאלא#FkFL | זאת הצורה |
| geometry/geometrician | ח.כ.מ./חכמי השעור | term | ספר_יסודי_התבונה_ומגדל_האמונה#Rqdk | חכמי השעור |
| geometry/geometrician | ח.כ.מ./חכמי המדות | term | Anonymous#3f0H | חכמי המדות |
| geometry/geometrician | ח.כ.מ./חכמי הגימטריא | term | תחבולות_המספר#zYpS | חכמי הגימטריאה |
| geometry/geometrician | ח.כ.מ./חכמי הגימטריא | term | חשבון_השטחים#HDsY | חכמי הגימאטריאה |
| geometry | הנדסה | term | קצור_המספר#oKmZ | חכמת ההנדסה |
| geometry | גיאומטריה | term | ספר_יסודי_התבונה_ומגדל_האמונה#WvOr | גיאומטריא |
| geometry | גימטריא | term | ספר_ג'יבלי_אלמוקבאלא#zdcI | גימטריא |
| geometry | ש.ע.ר./שעור | definition | ספר_יסודי_התבונה_ומגדל_האמונה#QN53 | geometry is a science that explains the measures and announces their value, their creation, their types, and their signs. חכמת השיעור היא חכמת מפרשת את הגדלים ומודיעה ערכם ויצירתם ומיניהן וסימניהן |
| geometry | נ.פ.ל./נפל | to be situated | חשבון_השטחים#JY7P | נפלה |
| geometry | נ.פ.ל./נפל | to be situated | חשבון_השטחים#lEvA | תפול |
| geometry | גימטריא | term | חשבון_השטחים#Grro | גימטריא |
| geometry | ש.ע.ר./שעור | term | ספר_יסודי_התבונה_ומגדל_האמונה#7kbk | חכמת השיעור |
| geometry | term | ספר_יסודי_התבונה_ומגדל_האמונה#tPav | אלהנדסה | |
| geometry | נ.פ.ל./נפל | to be situated | תחבולות_המספר#azrN | נופלת |
| geometry | נ.פ.ל./נפל | to be situated | תחבולות_המספר#RPnh | תפול |
| geometry | הנדסה | term | מלאכת_המספר#PJSB | הנדסה |
| geometry | ש.ע.ר./שעור | term | ספר_יסודי_התבונה_ומגדל_האמונה#yCv9 | חכמת השעור |
| geometry | גיאומטריה | term | ספר_יסודי_התבונה_ומגדל_האמונה#Sgh4 | יומטריא |
| geometry | מ.ד.ד./מדות | term | ספר_המספר_/_אליהו_מזרחי#cAbD | מדות |
| geometry | מ.ד.ד./מדידה | term | משנת_המדות#LghN | מדידה |
| geometry | מ.ד.ד./מדות | term | ספר_המספר_/_אליהו_מזרחי#NXxm | מלאכת המדות |
| geometry | מ.ד.ד./מדידה | term | ספר_המספר_/_אליהו_מזרחי#JOJz | מדידה |
| geometry | הנדסה | term | ספר_המספר_/_אליהו_מזרחי#JyR3 | הנדסה |
| geometry | הנדסה | term | ספר_המספר_/_אליהו_מזרחי#WrQe | חכמת ההנדסה |
| geometry | הנדסה | term | ספר_המספר_/_אליהו_מזרחי#TWz4 | הנדסא |
| geometry | הנדסה | term | מלאכת_המספר#1nMw | מלאכת ההנדסא |
| geometry | הנדסה | term | ספר_יסודי_התבונה_ומגדל_האמונה#vDEW | הנדסה |
| surface/gnomon | ר.ש.מ./רושם | term | ספר_האלזיברא#9D4Q | רושם התמונה |
| semicircle/greater than a semicircle | י.ת.ר./יתר | term | משנת_המדות#QxiL | יתרה |
| semicircle/greater than a semicircle | י.ת.ר./יתר | definition | משנת_המדות#Mepo | The surplus segment is any segment that is greater than a semicircle. והיתרה כל שהיא עודפת על חצי העגולה |
| line/height | ג.ב.ה./גובה | term | קצור_המספר#2ZCt | גובהו |
| line/height | ק.ו.מ./קומה | term | משנת_המדות#bcMz | קומה |
| line/height | ג.ב.ה./גובה | term | מלאכת_המספר#68a5 | גובה |
| line/height | ע.מ.ד./עמוד | term | ספר_הכללים_במספר#N2oI | עמודה |
| line/height | ע.מ.ד./עמוד | definition | משנת_המדות#ZKdD | The height is a perpendicular line descending from between the two sides to the base, and it is angled to the walls of the temple. והעמוד זה {{#annot:term|592,2289|H0NU}}חוט{{#annotend:H0NU}} הכולל היורד מבין שני הצלעים לקבע והוא בזוית למקצעות המשכן |
| line/height | ג.ב.ה./גובה | term | מלאכת_המספר#w6qQ | גובה |
| line/height | ג.ב.ה./גובה | term | קצור_המספר#Z57h | גובה |
| line/height | ג.ב.ה./גובה | term | בר_נותן_טעם#B53n | גובה |
| line/height | ע.מ.ד./עמוד | term | ספר_הכללים_במספר#iYwS | עמוד |
| line/height | ע.מ.ד./עמוד | term | משנת_המדות#1RG2 | עמוד |
| sphere/hemisheper | ת.ל.ל./תלול | term | משנת_המדות#zJmF | תלולה |
| polygon/hexagon | ש.ש.י./משושה | term | ספר_יסודי_התבונה_ומגדל_האמונה#19s3 | משושש |
| line/hypotenuse | ק.ב.ע./קבע | term | משנת_המדות#AnyJ | קבע |
| line/hypotenuse | י.ת.ר./יתר | term | קצור_המספר#MTF2 | יתר |
| line/hypotenuse | אלכסון | term | ספר_הכללים_במספר#QiEw | אלכסון |
| straight line/in a straight manner | י.ש.ר./על יושר | term | תחבולות_המספר#PN28 | על יושר |
| straight line/in a straight manner | י.ש.ר./ביושר | term | תחבולות_המספר#cDgm | ביושר |
| straight line/in a straight manner | י.ש.ר./ביושר | term | תחבולות_המספר#hJu6 | ביושר |
| straight line/in a straight manner | י.ש.ר./ביושר | term | חשבון_השטחים#VB0U | ביושר |
| straight line/in a straight manner | י.ש.ר./על יושר | term | ספר_האלזיברא#6UED | על יושר |
| straight line/in a straight manner | י.ש.ר./על יושר | term | חשבון_השטחים#mR21 | על יושר |
| geometric relations/inscribed | ר.ש.מ./מורשם | definition | ספר_היסודות_לאקלידס#A5Vw | The figure is said to be inscribed in a figure when each of its angles touches each of the respective sides of the figure in which it is inscribed. יאמר כי התמונה מורשמת בתמונה כאשר תהיה כל אחת מזויותיה ממששת לכל אחת מצלעות התמונה אשר נרשמת בה |
| surface/isosceles | ש.ו.ה./שוה צלעות | triangle | מלאכת_המספר#3Lky | משולש שוה הצלעות |
| surface/isosceles | ש.ו.ה./שוה | trapezoid | ספר_הכללים_במספר#s0uE | קטומת הראש שוה |
| surface/isosceles | ש.ו.ה./שוה שוקים | triangle | ספר_הכללים_במספר#SINi | משולש שוה השוקים |
| to join/joined | ד.ב.ק./דובק | term | ספר_ג'יבלי_אלמוקבאלא#lkPU | מדובקים ל |
| to join/joined | ד.ב.ק./דבק | term | תחבולות_המספר#FCgj | דבק |
| to join/joined | ד.ב.ק./דובק | term | תחבולות_המספר#pXGN | מדובקים |
| linear dimension/length | א.ר.כ./אורך | term | משנת_המדות#SQto | ארך |
| linear dimension/length | א.ר.כ./אורך | term | תחבולות_המספר#dIuF | ארכו |
| linear dimension/length | א.ר.כ./אורך | term | חשבון_השטחים#opY6 | אורכו |
| linear dimension/length | א.ר.כ./אורך | term | מלאכת_המספר#WuCf | אורך |
| linear dimension/length | א.ר.כ./אורך | term | חשבון_השטחים#CNQz | אורך |
| linear dimension/length | term | ספר_המספר_/_אברהם_אבן_עזרא#tTbn | ארכו | |
| linear dimension/length | א.ר.כ./אורך | term | ספר_האלזיברא#xJSR | ארכו |
| linear dimension/length | א.ר.כ./אורך | term | בר_נותן_טעם#wbTk | אורך |
| linear dimension/length | א.ר.כ./אורך | term | בר_נותן_טעם#x8jj | ארך |
| linear dimension/length | א.ר.כ./אורך | term | מלאכת_המספר#h6f3 | אורך |
| linear dimension/length | א.ר.כ./אורך | term | תחבולות_המספר#vaVj | אורך |
| semicircle/less than a semicircle | ח.ס.ר./חסר | definition | משנת_המדות#qLjq | The deficient segment is any segment that is smaller than a semicircle. החסרה כל שהיא פחותה מחצי העגולה |
| semicircle/less than a semicircle | ח.ס.ר./חסר | term | משנת_המדות#ZNjf | חסרה |
| geometrical shape/line | מ.ש.כ./משוך | term | משנת_המדות#aohw | משוכים |
| geometrical shape/line | ק.ו.י./קו | definition | ספר_היסודות_לאקלידס#wN6J | The '''line''' is a length that has no breadth. והקו הוא אורך אין רוחב לו |
| geometrical shape/line | ק.ו.י./קו | term | קצור_המספר#NdeC | קו |
| geometrical shape/line | חוט | term | משנת_המדות#H0NU | חוט |
| geometrical shape/line | ק.ו.י./קו | term | תחבולות_המספר#XNSA | קו |
| geometrical shape/line | ק.ו.י./קו | term | ספר_המספר_/_אליהו_מזרחי#zEkb | קוים |
| geometrical shape/line | ק.ו.י./קו | term | האריתמטיקה_של_ניקומכוס#RC38 | קו |
| geometrical shape/line | ק.ו.י./קו | term | ספר_המספר_/_אליהו_מזרחי#2j7U | קו |
| geometrical shape/line | ק.ו.י./קו | term | ספר_האלזיברא#n2q8 | קו |
| geometrical shape/line | ק.ו.י./קו | definition | האריתמטיקה_של_ניקומכוס#3xJt | The line is that which has only length, and that which has only length is a line. והקו מה שהיה לו אורך לבד ומה שהיה לו אורך לבד הוא קו |
| geometrical shape/line | ק.ו.י./קו | term | חשבון_השטחים#ZCVZ | קו |
| geometrical shape/line | ק.ו.י./קו | term | חשבון_השטחים#gKGj | קוים |
| geometrical shape/line | ק.ו.י./קו | term | ספר_מעשה_חושב#9DYp | קוים |
| geometrical shape/line | ק.ו.י./קו | definition | מלאכת_המספר#wrX5 | the line is a quantity of length without breadth and depth, whose ends are two points. והקו הוא כמות אורך בלתי רוחב ועומק וקצותיו הם ב' נקודות |
| geometrical shape/line | ק.ו.י./קו | term | ספר_מעשה_חושב#jwYt | קו |
| geometrical shape/line | ק.ו.י./קו | term | תחבולות_המספר#1nUp | קוים |
| geometrical shape/line | ק.ו.י./קו | definition | האריתמטיקה_של_ניקומכוס#mITP | The line has one dimension. והקו בעל משך אחד |
| geometrical shape/line | מ.ש.כ./משוך | term | משנת_המדות#3VKI | המשוך |
| geometry/linear dimension | ר.ח.ק./מרחק | definition | ספר_יסודי_התבונה_ומגדל_האמונה#ehyj | the dimensions are three: length, breadth and depth, or height. והמרחקים הם ג' אורך ורחב ועומק או רום |
| geometry/linear dimension | ר.ח.ק./מרחק | term | מלאכת_המספר#0yxe | מרחקיו |
| geometry/linear dimension | ר.ח.ק./מרחק | term | מלאכת_המספר#sNrA | מרחקי' |
| angle/obtuse angle | ר.ו.ח./נרוח | term | ספר_יסודי_התבונה_ומגדל_האמונה#LHoW | זוית נרוחת |
| angle/obtuse angle | ר.ו.ח./נרוח | definition | ספר_יסודי_התבונה_ומגדל_האמונה#XQbW | the obtuse angle exceeds over the right [angle] in its value. והזוית הנרוחת היא המוסיפה בערכה על הנצבת |
| angle/obtuse angle | ר.ו.ח./נרוח | definition | ספר_היסודות_לאקלידס#szjV | The greater than a right angle is called an '''obtuse angle'''. ואשר היא גדולה מנצבת תקרא נרוחת |
| angle/obtuse angle | כ.ס.ח./כסוחה | term | משנת_המדות#hYTA | כסוחה |
| angle/obtuse angle | ר.ח.ב./רחב | term | משנת_המדות#z0OW | זויות רחבים |
| angle/obtuse angle | ר.ח.ב./רחב | term | משנת_המדות#lTJw | רחבה |
| obtuse angle/obtuse-angled | ר.ו.ח./מרוח | triangle-definition | ספר_יסודי_התבונה_ומגדל_האמונה#7MuR | the third species is that which has one obtuse angle, which is called an obtuse-angled [triangle]. והמין השלישי הוא אשר יש לו זוית אחת נרוחת והוא נקרא מרווח הזוית |
| obtuse angle/obtuse-angled | ר.ו.ח./מרוח | triangle | ספר_הכללים_במספר#iUfN | משולש מרויח הזויות |
| obtuse angle/obtuse-angled | ר.ו.ח./מרוח | triangle | ספר_יסודי_התבונה_ומגדל_האמונה#dxct | המשלש מרווח הזוית |
| obtuse angle/obtuse-angled | מ.ו.ט./מתמוטטת | trapezoid | ספר_הכללים_במספר#Bzov | מתמוטטת |
| obtuse angle/obtuse-angled | ר.ו.ח./נרוח | triangle-definition | ספר_היסודות_לאקלידס#g9wm | The '''obtuse-angled triangle''' is that which has an obtuse angle. והמשולש הנרוח הזוית והוא אשר לו זוית נרוחת |
| obtuse angle/obtuse-angled | מ.ו.ט./מתמוטטת | trapezoid | ספר_הכללים_במספר#xzTs | קטומה מתמוטטת |
| obtuse angle/obtuse-angled | פ.ס.ח./פסוחה | triangle | משנת_המדות#uuSe | פסוחה |
| line/parallel | נ.כ.ח./נכחי | term | חשבון_השטחים#HjSC | נכחי ל |
| line/parallel | נ.כ.ח./נכחי | term | ספר_הכללים_במספר#uvqN | נכוחיים |
| line/parallel | נ.כ.ח./נכחי | term | ספר_הכללים_במספר#E7Zk | נכוחית |
| line/parallel | נ.כ.ח./נכחי | term | תחבולות_המספר#LCaE | נכחי ל |
| line/parallel | נ.כ.ח./נכחי | term | חשבון_השטחים#cvrF | נכחיים ל |
| line/parallel | נ.כ.ח./נכחי | term | תחבולות_המספר#Ycx6 | נכחיים ל |
| line/parallel | נ.כ.ח./על נכוחות | term | תחבולות_המספר#zRs4 | על נכוחות |
| line/parallel | נ.כ.ח./נכחי | definition | ספר_היסודות_לאקלידס#Zac6 | The '''parallel straight lines''' are those that are in the same plane such that if they are drawn endlessly in both directions, they do not meet one another in either direction. והקוים הישרים הנכחיים הם אשר יהיו על שטח אחד שוה ואם הוצאו בשני הצדדים אל לא תכלית לא יפגשו באחד מהם |
| line/parallel | נ.כ.ח./נכחי | term | ספר_הכללים_במספר#Jegs | נכוחי |
| line/parallel | נ.כ.ח./על נכוחות | term | חשבון_השטחים#w6CY | על נכחות |
| line/parallel | נ.כ.ח./על נכוחות | term | תחבולות_המספר#a4Jr | על נכוחות |
| line/parallel | נ.כ.ח./נכחי | term | ספר_ג'יבלי_אלמוקבאלא#lKms | נכחיים |
| line/parallel | נ.כ.ח./על נכוחות | term | חשבון_השטחים#5Sdc | על נכחות |
| line/parallel | נ.כ.ח./נכחי | term | ספר_האלזיברא#cNKO | נכוחי ל |
| line/parallel | נ.כ.ח./נכחי | term | ספר_ג'יבלי_אלמוקבאלא#dReT | נכחי ל |
| line/parallel | נ.כ.ח./נכחי | term | ספר_האלזיברא#W8aP | נכחי לקו |
| solid/parallelepiped | נ.כ.ח./נכחי השטחים | term | ספר_היסודות_לאקלידס#w1JC | מוגשם נכחיי השטחים |
| solid/parallelepiped | נ.כ.ח./נכחי השטחים | term | ספר_היסודות_לאקלידס#5wo6 | מוגשמי כ"ב וז"ל נכחי השטחים |
| quadrilateral/parallelogram | ע.י.נ./דומה למעוין | definition | ספר_יסודי_התבונה_ומגדל_האמונה#Di4Q | the fourth [category] is similar to the second whose two of its sides that are parallel to each other, only that its angles are not right [angles] - this is called a parallelogram. והרביעי דומה לשני שכל שני מצלעותיו העומדות זו כנגד זו נכחיות ושוות אלא שזויותיו אינן נצבות וזה נקרא דומה למעויין |
| quadrilateral/parallelogram | ע.י.נ./דומה למעוין | definition | ספר_היסודות_לאקלידס#Wn4u | The '''rhomboid''' is that whose opposite sides are equal to one another but is neither equilateral nor right-angled. ומהם הדומה למעויין והוא אשר כל שתי צלעות ממנו שזו כנגד זו שוות ואינו שוה הצלעות ואינו נצב הזויות |
| quadrilateral/parallelogram | נ.כ.ח./נכחי הצלעות | term | ספר_היסודות_לאקלידס#9qdL | השטחים נכחיי הצלעות |
| quadrilateral/parallelogram | נ.כ.ח./נכחי הצלעות | term | ספר_היסודות_לאקלידס#ERBz | נכחי הצלעות |
| polygon/pentagon | ח.מ.ש./מחומש | term | ספר_יסודי_התבונה_ומגדל_האמונה#rQuH | מחומשות |
| polygon/pentagon | ח.מ.ש./מחומש | term | ספר_יסודי_התבונה_ומגדל_האמונה#w5N9 | המחומש |
| polygon/pentagon | ח.מ.ש./מחומש | term | האריתמטיקה_של_ניקומכוס#m2Dh | מחומש |
| line/perimeter | ס.ב.ב./סביבה | definition | משנת_המדות#AFu9 | What is the perimeter? it is the line that circumscribes the circle, as it is said: ''and a line of thirty cubits did compass it round about'' [Chronicles 2, 4, 2]. איזו היא סביבה הוא הקו המקיף את העגול שנ' ''וקו שלשים באמה יסב אותו סביב'' דברי הימים ב, ד, ב |
| line/perimeter | נ.ק.פ./הקף | term | מלאכת_המספר#ngFU | הקף העגולה |
| line/perimeter | term | ספר_המספר_/_אברהם_אבן_עזרא#Qhlj | הקו הסובב | |
| line/perimeter | כמות | term | מלאכת_המספר#RwcD | כמות העגולה |
| line/perimeter | term | ספר_המספר_/_אברהם_אבן_עזרא#kQKO | קו העגול | |
| line/perimeter | ס.ב.ב./סביבה | term | משנת_המדות#ktSB | סביבה |
| line/perpendicular | ע.מ.ד./מעומד | term | מלאכת_המספר#aMf5 | מעומד |
| line/perpendicular | ק.צ.ר./קצור | term | משנת_המדות#3vSq | קצורים |
| line/perpendicular | ע.מ.ד./מעומד | term | מלאכת_המספר#GhS0 | מעומד |
| surface/planar | י.ש.ר./ישר | term | ספר_יסודי_התבונה_ומגדל_האמונה#JAjC | ישר |
| surface/planar | ש.ט.ח./שטוח | term | ספר_המספר_/_אליהו_מזרחי#5c6J | שטוחות |
| surface/planar | מ.צ.ע./ממוצע | term | משנת_המדות#epCp | ממוצע |
| angle/plane angle | פ.ש.ט./פשוט | definition | ספר_היסודות_לאקלידס#YkbQ | The '''plane angle''' is the inclination of two given lines to one another in a plane, so that they meet one another not in a straight line. והזוית הפשוטה היא נטיית כל אחד משני קוים מונחים בשטח שוה מדובקים על בלתי יושר האחד מן האחר |
| angle/plane angle | ש.ט.ח./שטוחה | term | ספר_יסודי_התבונה_ומגדל_האמונה#5Ggu | זוית שטוחה |
| geometrical shape/point | נ.ק.ד./נקודה | term | תחבולות_המספר#HGlB | נקודות |
| geometrical shape/point | נ.ק.ד./נקודה | term | ספר_האלזיברא#oWim | נקדת |
| geometrical shape/point | נ.ק.ד./נקודה | definition | ספר_היסודות_לאקלידס#I0Aq | The '''point''' is a thing that has no part. |style="width:45%;text-align:right;"|הנקודה היא דבר אין לה חלק ולא הנחה |
| geometrical shape/point | נ.ק.ד./נקודה | term | ספר_יסודי_התבונה_ומגדל_האמונה#g5iF | נקדה |
| geometrical shape/point | midpoint | ספר_המספר_/_אברהם_אבן_עזרא#vdqX | נקודה | |
| geometrical shape/point | נ.ק.ד./נקודה | term | האריתמטיקה_של_ניקומכוס#Qk2e | נקודה |
| geometrical shape/point | נ.ק.ד./נקודה | term | חשבון_השטחים#G3Un | נקודת |
| geometrical shape/point | נ.ק.ד./נקודת חלוק | midpoint | מלאכת_המספר#3Tls | נקודת החלוק |
| geometrical shape/point | נ.ק.ד./נקודה | term | קצור_המספר#UMxT | נקודה |
| geometrical shape/point | נ.ק.ד./נקודה | term | תחבולות_המספר#eSZc | נקדת |
| geometrical shape/point | נ.ק.ד./נקודת חלוקה | midpoint | מלאכת_המספר#BIOa | נקודת החלוקה |
| geometrical shape/point | נ.ק.ד./נקודה | term | האריתמטיקה_של_ניקומכוס#u4ba | נקודות |
| axis/pole | ק.ט.ב./קוטב | definition | ספר_יסודי_התבונה_ומגדל_האמונה#TU07 | the two points that are the ends of the axis are called the beginnings of the axis and in Arabic they are called the poles of the axis. וב' הנקודות אשר הם תכליתי הבריח נקראים ראשי הבריח וקוראים להם בלשון ישמעאל קטבי הבריח |
| surface/polygon | י.ש.ר./ישר קוים | term | האריתמטיקה_של_ניקומכוס#SU8c | השטחים ישרי הקוים |
| surface/polygon | י.ש.ר./ישר | term | האריתמטיקה_של_ניקומכוס#9SO5 | השטחים הישרים |
| surface/polygon | definition | ספר_יסודי_התבונה_ומגדל_האמונה#K7RF | the third is the polygon that the lines surrounding it exceed four. והשלישי הוא המרבה דפנות אשר הקוים המקיפים אותו הן עודפים על ד' | |
| surface/polygon | ר.ב.ה./מרבה צלעות | term | ספר_יסודי_התבונה_ומגדל_האמונה#xQVA | השטח המרבה צלעות |
| solid/prism | ח.מ.ש./מחומש | pentagonal | ספר_הכללים_במספר#K9aE | מצבה מחומשת |
| solid/prism | ג.ר.ר./מתגורר | term | האנציקלופדיה_של_אבו_אלצלת#aqI8 | מתגורר |
| solid/prism | י.צ.ב./מצבה | term | ספר_הכללים_במספר#NojB | מצבה |
| solid/prism | י.צ.ב./מצבה | term | ספר_יסודי_התבונה_ומגדל_האמונה#nZFZ | מצבה |
| solid/prism | ג.ר.ר./מגורר | term | האנציקלופדיה_של_אבו_אלצלת#9vMo | מגורר |
| solid/prism | ע.מ.ד./עמוד | term | משנת_המדות#kXIU | עמוד |
| solid/prism | ג.ר.ר./מגורר | triangular-definition | ספר_היסודות_לאקלידס#Dfy8 | A prism is a solid figure contained by three rectangles and two triangles. התמונה המוגשמת המגוררת היא אשר יקיפו בה שלשה שטחים נכחיי הצלעות ושני שטחים משולשים |
| solid/prism | י.צ.ב./מצבה | term | ספר_יסודי_התבונה_ומגדל_האמונה#6azb | מצבות |
| solid/prism | ח.צ.י./חצויה | triangular | ספר_הכללים_במספר#FrRB | מצבה חצוית הראש |
| solid/prism | ח.צ.י./חצויה | triangular | ספר_הכללים_במספר#rw6P | מצבה משולשת חצויה |
| solid/prism | ח.צ.י./חצויה | triangular | ספר_הכללים_במספר#OosO | מצבה חצויה |
| solid/prism | ר.ב.ע./מרובע | square | משנת_המדות#aQuk | עמוד מרבע |
| solid/pyramid | ז.נ.ב./מזונב | term | האריתמטיקה_של_ניקומכוס#Ofm0 | מזונב |
| solid/pyramid | ח.ד.ד./מחודד | triangular | האריתמטיקה_של_ניקומכוס#xeb3 | המשולש המוגשם המחודד |
| solid/pyramid | מ.ש.כ./משוך | term | משנת_המדות#JO3X | משוך |
| solid/pyramid | ח.ד.ד./מחודד | term | האנציקלופדיה_של_אבו_אלצלת#Lvtr | מחדדים |
| solid/pyramid | ח.ד.ד./מחודד | term | מלאכת_המספר#NwMW | מחודד |
| solid/pyramid | ש.ל.ש./משולש | triangular | האריתמטיקה_של_ניקומכוס#Gr1Y | הגרם המשולש |
| solid/pyramid | ח.ד.ד./מחודד | term | האריתמטיקה_של_ניקומכוס#pjvt | המוגשם המחודד |
| solid/pyramid | ז.נ.ב./מזונב | term | האריתמטיקה_של_ניקומכוס#gLyL | מזונבים |
| solid/pyramid | ח.ד.ד./מחודד | term | האריתמטיקה_של_ניקומכוס#6x7Y | מחודדים |
| solid/pyramid | ח.ד.ד./מחודד | term | האריתמטיקה_של_ניקומכוס#CG7S | מחודד |
| solid/pyramid | ז.נ.ב./מזדנב | term | האריתמטיקה_של_ניקומכוס#o7LK | גרם מזדנב |
| surface/quadrilateral | term | תחבולות_המספר#W9nk | שטח הכאת | |
| surface/quadrilateral | ר.ב.ע./מרובע | term | קצור_המספר#0nqL | מרובע |
| surface/quadrilateral | ש.ט.ח./מושטח | term | קצור_המספר#i6VS | מושטח |
| surface/quadrilateral | ר.ב.ע./מרובע | term | משנת_המדות#4N37 | מרבעת |
| surface/quadrilateral | ר.ב.ע./מרובע | definition | ספר_יסודי_התבונה_ומגדל_האמונה#bfge | the second is the quadrilateral which is the surrounded by four straight lines. והשני הוא המרובע והוא אשר יקיף אותו ד' קוים ישרים |
| surface/quadrilateral | ר.ב.ע./מרובע | term | מלאכת_המספר#Vy5I | מרובע |
| surface/quadrilateral | ש.ט.ח./מושטח | term | קצור_המספר#3lah | מושטח |
| surface/quadrilateral | ר.ב.ע./מרובע | term | בר_נותן_טעם#LjAq | מרובע |
| quadrilateral/rectangle | טבלה | term | משנת_המדות#wrZo | טבלא |
| quadrilateral/rectangle | ר.ב.ע./מרובע ארוך | term | ספר_הכללים_במספר#euTK | מרובע ארוך |
| quadrilateral/rectangle | ח.ל.פ./מתחלף ארכים | definition | ספר_היסודות_לאקלידס#bvdV | The '''oblong''' is that which is right-angled but not equilateral. ומהם המתחלף הארכים והוא הנצב הזויות לא שוה הצלעות |
| quadrilateral/rectangle | ר.ב.ע./מרובע ארוך | term | ספר_הכללים_במספר#KIEb | מרובע ארוך |
| quadrilateral/rectangle | ש.ט.ח./שטח נצב הזויות | term | ספר_היסודות_לאקלידס#UxZC | שטח הנצב הזויות |
| quadrilateral/rectangle | ש.ט.ח./שטח נצב הזויות | term | ספר_היסודות_לאקלידס#PIuX | שטח נצב הזויות |
| quadrilateral/rectangle | ש.ט.ח./שטח נצב הזויות | term | תחבולות_המספר#tLXb | שטח נצב הזויות |
| quadrilateral/rectangle | ש.ט.ח./שטח נצב הזויות | term | ספר_האלזיברא#vcTz | שטח נצב הזויות |
| quadrilateral/rectangle | ש.ט.ח./שטח נצב הזויות | term | ספר_האלזיברא#oh24 | השטח הנצב הזויות |
| quadrilateral/rectangle | ר.ב.ע./מרובע ארוך | definition | ספר_יסודי_התבונה_ומגדל_האמונה#Ig6X | the second [category] is that whose two of its sides are parallel and equal, its [two] other sides are also equal, and so are all of its four angles - this is called a rectangle. והשני הוא אשר שני מצלעיו הם נכוחיות ושוות וכן צלעותיו אחרות שוות כמו כן וד' זויותיו כלם וזה נקרא מרובע ארוך |
| quadrilateral/rhombus | ע.י.נ./מעוין | definition | ספר_יסודי_התבונה_ומגדל_האמונה#ylyu | the third [category] is similar to the first in its sides that are equal, only that its angles are not right [angles] - this is called a rhombus. והשלישי דומה לראשון בצלעיו שהן שוות אלא שזויותיו אינם נצבות וזה נקרא מעויין |
| quadrilateral/rhombus | ע.י.נ./מעוין | term | ספר_הכללים_במספר#gBop | מעויין |
| quadrilateral/rhombus | ע.י.נ./מעוין | term | ספר_הכללים_במספר#6VRn | מרובע המעויין |
| quadrilateral/rhombus | ע.י.נ./מעוין | definition | ספר_היסודות_לאקלידס#us1T | The '''rhombus''' is that which is equilateral but not right-angled. ומהם המעויין והוא השוה הצלעות ואינו נצב הזויות |
| angle/right angle | י.צ.ב./נצב | term | ספר_המספר_/_אליהו_מזרחי#4KlC | זויות נצבות |
| angle/right angle | י.צ.ב./נצב | term | ספר_יסודי_התבונה_ומגדל_האמונה#g94r | זוית נצבת |
| angle/right angle | י.צ.ב./נצב | term | תחבולות_המספר#kliQ | זוית נצבה |
| angle/right angle | י.צ.ב./נצב | term | משנת_המדות#w2f4 | נצבה |
| angle/right angle | י.צ.ב./נצב | term | מלאכת_המספר#u7EE | זויות נצבות |
| angle/right angle | י.צ.ב./נצב | term | קצור_המספר#dSxJ | הזוית הנצבה |
| angle/right angle | י.צ.ב./נצב | definition | ספר_יסודי_התבונה_ומגדל_האמונה#9aNz | The right angle is one of two angles which is on the sides of a line that is perpendicular to another line and each is equal to the other in value. והזוית נצבה היא אחת מב' זויות אשר על צדי קו העומד על קו אחר וכל אחת מהן שוה בערך לחברתה |
| right angle/right-angled | י.צ.ב./נצב | triangle | ספר_יסודי_התבונה_ומגדל_האמונה#syPT | נצב הזויות |
| right angle/right-angled | י.צ.ב./נצב | triangle-definition | ספר_יסודי_התבונה_ומגדל_האמונה#KjlN | the second species is that which has one angle that is right and two [angles] that are acute - this is called a right-angled triangle. והמין השני הוא אשר יש לו זוית אחת נצבת ושתים חדות והוא נקרא משלש נצב הזוית |
| right angle/right-angled | י.צ.ב./נצב | triangle | ספר_הכללים_במספר#c8w5 | משולש נצב הזויות |
| right angle/right-angled | י.צ.ב./נצב | triangle | ספר_הכללים_במספר#YJQE | משולש נצב הזוית |
| right angle/right-angled | י.צ.ב./נצב | triangle | ספר_הכללים_במספר#1xD2 | משולשים מוצבי זוית |
| right angle/right-angled | י.צ.ב./נצב | triangle-definition | ספר_היסודות_לאקלידס#BAtH | The '''right-angled triangle''' is that which has a right angle. המשולש נצב הזוית והוא אשר לו זוית נצבת |
| right angle/right-angled | י.צ.ב./נצב | triangle | משנת_המדות#aKHk | נצבה |
| surface/scalene | ח.ל.פ./חלוף | triangle | משנת_המדות#ADtV | משלשת חלופים |
| surface/scalene | ח.ל.פ./חלופה | trapezoid | ספר_הכללים_במספר#oONP | קטומת הראש חלופה |
| surface/scalene | ח.ל.פ./חלוף | triangle | משנת_המדות#O4ly | חלופים |
| surface/scalene | פ.ת.ל./נפתל | quadrilateral | ספר_יסודי_התבונה_ומגדל_האמונה#BUOn | מרובע נפתל |
| surface/scalene | ש.נ.י./משונה | quadrilateral | משנת_המדות#Tcr7 | המרבעות המשונות |
| surface/scalene | ח.ל.פ./מתחלף צלעות | triangle | ספר_הכללים_במספר#F605 | משולש מתחלף |
| surface/scalene | ח.ל.פ./מתחלף צלעות | triangle | מלאכת_המספר#sAxB | משולש מתחלף הצלעות |
| surface/scalene | ח.ל.פ./מתחלף צלעות | triangle | ספר_הכללים_במספר#Hcvc | משולש מתחלף הצלעות |
| surface/scalene | ש.נ.י./משונה | quadrilateral | משנת_המדות#rF67 | משונה |
| geometry/section | ק.ט.פ./קטופה | term | משנת_המדות#mCQR | קטופה |
| circle/sector | ח.ת.כ./חתוך | definition | ספר_היסודות_לאקלידס#s56d | A sector of a circle is a shape that is contained by two straight lines containing an angle at the center of the circle, and the arc that is cut off from the circle by these two lines. י וחתוך העגולה היא תמונה אשר יקיפו בה שני קוים ישרים יקיפו בזוית על מרכז העגולה וקשת יקיפוה אותם שני הקוים מן העגולה |
| line/segment | ח.ל.ק./חלק | term | חשבון_השטחים#B51m | חלקים |
| line/segment | ח.ל.ק./חלק | term | חשבון_השטחים#QXND | חלקיו |
| line/segment | ח.ל.ק./חלק | term | תחבולות_המספר#8rKf | חלקים |
| line/segment | ח.ל.ק./חלק | term | ספר_האלזיברא#GSR6 | חלק |
| line/segment | פ.ס.ק./פסקה | term | משנת_המדות#hGWl | פסקאות |
| circle/segment of a circle | ח.ת.כ./חתיכה | definition | ספר_היסודות_לאקלידס#XaeS | The '''segment of the circle''' is the figure contained by a straight line and an arc on the circumference that is either smaller or greater than its half. וחתיכת העגול היא תמונה יקיפו בה קו ישר וקשת ממקיף העגולה אם קטנה מחציה או גדולה |
| circle/segment of a circle | ק.ש.ת./קשותה | term | משנת_המדות#qGNQ | קשותה |
| circle/segment of a circle | ח.ת.כ./חתיכה | definition | ספר_היסודות_לאקלידס#ISnG | A segment of a circle is that which is contained by a straight line that is called a chord and the segment of circumference that is called an arc. ו חתיכת העגולה היא אשר יקיף בה קו ישר יקרא המיתר והחלק מן הקו המקיף יקרא הקשת |
| circle/semicircle | י.ש.ר./ישרה | term | משנת_המדות#aGCk | ישרה |
| circle/semicircle | י.ש.ר./ישרה | definition | משנת_המדות#YhPn | Which is the semicircle? Any segment that is half a circle, no more and no less. איזו היא ישרה כל שהיא עומדת בחצי העגולה לא חסר ולא יתר |
| solid/sharp solid | ל.ה.ב./מתלהב | term | האנציקלופדיה_של_אבו_אלצלת#Pmc5 | מתלהבים |
| surface/side | צלע | definition | משנת_המדות#IL6w | What is the side? it is the holder of the surface's walls, as it is said: ''the altar shall be square''. אי זו היא הצלע זה המחזיק דופנותיו של גג שנ' ''רבוע יהיה המזבח''שמות כז, א |
| surface/side | צלע | term | עיר_סיחון#YlsD | צלעות |
| surface/side | צלע | term | מלאכת_המספר#f9Sc | צלעות |
| surface/side | צלע | term | קצור_המספר#t24N | צלע |
| surface/side | term | ספר_המספר_/_אברהם_אבן_עזרא#ZJhZ | פאת | |
| surface/side | צלע | term | חשבון_השטחים#CK5Q | צלעיו |
| surface/side | י.ש.ב./תושבת | term | ספר_המספר_/_אליהו_מזרחי#SLbt | תושבות |
| surface/side | צלע | term | תחבולות_המספר#DtxV | צלע |
| surface/side | צלע | term | ספר_האלזיברא#JpoA | צלע |
| surface/side | צלע | term | קצור_המספר#z4ec | צלעות |
| surface/side | צלע | term | מלאכת_המספר#Rtkh | צלע |
| surface/side | צלע | term | משנת_המדות#bpGS | צלע |
| surface/side | צלע | term | ספר_האלזיברא#I58U | צלע מרבע |
| surface/side | צלע | term | תחבולות_המספר#lBcX | צלעיו |
| surface/side | צלע | term | חשבון_השטחים#P0If | צלע |
| geometric relations/similar figures | ד.מ.י./דומה | solids-definition | ספר_היסודות_לאקלידס#oKi8 | The similar solid shapes are those that each solid of which is contained by the same number of surfaces as the number of the surfaces containing the other, and each surface is similar to its corresponding surface in the other solid. התמונות המוגשמות הדומות הם אשר יקיף כל מוגשם מהם ממנין השטחים כמו מנין מה שיקיף באחר ויהיה כל שטח מאחד מהם דומה בשטח אשר הוא גילו מן המוגשם האחר ועל בריאתו |
| geometric relations/similar figures | surfaces-definition | ספר_היסודות_לאקלידס#7gj7 | The similar rectilinear figures are those whose angles are equal and whose sides about the equal angles are proportional. השטחים המתדמים הם אשר זויותיהם שוות וצלעותיהם המקיפות בזויות השוות מתיחסות | |
| geometrical shape/solid | ג.ר.מ./גרם | definition | האריתמטיקה_של_ניקומכוס#kMEJ | It was said that the solid adds a dimension of depth to the surface, since the surface has only length and breadth ואמרנו שהגרם הוא אשר יוסיף משך בעומק על השטח אחר שהשטח אורך ורחב לבד |
| geometrical shape/solid | ג.ש.מ./גשם | term | מלאכת_המספר#x4VO | גשם |
| geometrical shape/solid | ג.ש.מ./מוגשם | term | מלאכת_המספר#NWfq | מוגשם |
| geometrical shape/solid | גוף | term | משנת_המדות#lVlq | גוף |
| geometrical shape/solid | גו | term | משנת_המדות#livj | גוו |
| geometrical shape/solid | גג | term | משנת_המדות#NnXf | גג |
| geometrical shape/solid | ג.ש.מ./גשם | definition | מלאכת_המספר#LrBm | The solid is a quantity that has three dimensions, which are length, breadth and depth, whose limits are surfaces. הגשם הוא כמות שיש לו ג' {{#annot:term|315,1891|sNrA}}מרחקי'{{#annotend:sNrA}} שהם אורך ורוחב ועומק שתכליותיו הם ב' שטחי' |
| geometrical shape/solid | ג.ש.מ./גשם | term | מלאכת_המספר#CTjN | גשם |
| geometrical shape/solid | ג.ש.מ./גשם | term | האריתמטיקה_של_ניקומכוס#Q8TU | גשם |
| geometrical shape/solid | ג.ש.מ./גשם | definition | האריתמטיקה_של_ניקומכוס#nUtW | One may argue by saying in a general statement that any thing that has length, breadth, and depth is a solid, and every solid has length, breadth, and depth. ואולי יהיה לטוען שיאמר הנה במאמר כולל שכל בעל אורך ורוחב ועומק הוא גשם וכל גשם בעל אורך ורחב ועומק |
| geometrical shape/solid | ג.ש.מ./גשם | term | ספר_מעשה_חושב#RgAm | גשם |
| geometrical shape/solid | ג.ש.מ./גשם | definition | האריתמטיקה_של_ניקומכוס#Sy4l | The three-dimensional is the solid. ומה שהיה בעל שלשה המשכים הוא גשם |
| geometrical shape/solid | ג.ש.מ./גשם | term | בר_נותן_טעם#eHwo | גשם |
| geometrical shape/solid | ג.ר.מ./גרם | term | האריתמטיקה_של_ניקומכוס#Ff7g | גרמים |
| geometrical shape/solid | גוף | term | האריתמטיקה_של_ניקומכוס#3czY | גוף |
| geometrical shape/solid | ג.ר.מ./גרם | term | האריתמטיקה_של_ניקומכוס#rQJK | הגרם |
| geometrical shape/solid | ג.ל.מ./גולם | definition | ספר_יסודי_התבונה_ומגדל_האמונה#5tIP | The first is called solid, and it has three dimensions. הראשון נק' גולם ויש לו ג' מרחקים |
| geometrical shape/solid | ג.ל.מ./גולם | term | ספר_יסודי_התבונה_ומגדל_האמונה#xmto | גלם |
| geometrical shape/solid | ג.ל.מ./גולם | term | ספר_יסודי_התבונה_ומגדל_האמונה#jKvx | גולמים |
| geometrical shape/solid | ג.ש.מ./גשם | term | ספר_המספר_/_אליהו_מזרחי#4qT1 | גשמים |
| geometrical shape/solid | ג.ש.מ./גשם | term | ספר_המספר_/_אליהו_מזרחי#ZoVN | גשם |
| geometrical shape/solid | ג.ש.מ./מוגשם | term | ספר_המספר_/_אליהו_מזרחי#yKif | מוגשמות |
| geometrical shape/solid | ג.ל.מ./גולם | term | ספר_הכללים_במספר#5Z9l | גולם |
| geometrical shape/solid | ע.ק.ב./מעוקב | definition | Arithmetic_Textbook_by_R._Aharon_ben_Isaac#Z0L5 | מעוקב שהוא הגשם אשר ימצאו בו השלושה רחקים שהם אורך ורוחב ועומד |
| geometrical shape/solid | גוף | term | ספר_הכללים_במספר#EyrJ | גוף |
| solid/solid angle | קרן | term | ספר_יסודי_התבונה_ומגדל_האמונה#1mxy | קרנות |
| solid/solid angle | קרן | term | ספר_יסודי_התבונה_ומגדל_האמונה#2LQy | קרנות השטח |
| solid/solid angle | ג.ל.מ./גלומה | term | ספר_יסודי_התבונה_ומגדל_האמונה#JS01 | זוית גולמית |
| solid/solid angle | קרן | term | ספר_יסודי_התבונה_ומגדל_האמונה#SX31 | קרן |
| solid/sphere | כ.ד.ר./כדור | term | ספר_הכללים_במספר#MkFQ | כדור |
| solid/sphere | כ.ד.ר./כדור | definition | ספר_היסודות_לאקלידס#sI0K | The sphere is when the semicircle is drawn round with the diameter fixed in two points, so it does not move, and the arc, which is half the perimeter, revolves until it returns to its position; it is the circular solid. The center of the sphare and the center of the circle are the same. הכדור הוא מה שיעבור חצי עגולה כאשר יקוים קו הקוטר בין שני כשורים עד שלא יסור וסבבה הקשת אשר היא חצי הקו המקיף עד שתשוב אל מקומה והוא המוגשם העגול ומרכז הכדור ומרכז העגולה אחד |
| solid/sphere | כ.ד.ר./כדור | term | מלאכת_המספר#NYpt | כדור |
| solid/sphere | כ.ד.ר./כדור | definition | ספר_יסודי_התבונה_ומגדל_האמונה#MjPy | the solid that is surrounded by a circular surface is called sphere. והגולם אשר יקיף אותו פרוש אחד עגול הוא הנקרא כדור |
| solid/sphere | כ.ד.ר./כדור | definition | ספר_יסודי_התבונה_ומגדל_האמונה#WlA0 | It is that which is surrounded by one surface all around and at its center there is one point such that all the lines that are drawn from it to the surface of the sphere are equal to each other. הוא אשר יקיפו פרוש אחד מכל סביביו ובאמצעיתו נקודה אחת וכל הקוים היוצאים ממנה אל פרוש הכדור שוים זה לזה |
| solid/sphere | ת.ל.י./תלוי | term | משנת_המדות#uV4d | תלויה |
| solid/sphere | כ.ד.ר./כדור | term | ספר_יסודי_התבונה_ומגדל_האמונה#hzZC | כדור |
| quadrilateral/square | ש.ו.ה./שוה צלעות | term | ספר_ג'יבלי_אלמוקבאלא#0caF | שטח אחד שוה הצלעות |
| quadrilateral/square | ש.ו.ה./שוה צלעות | term | ספר_ג'יבלי_אלמוקבאלא#94Yd | מרובע שוה הצלעות |
| quadrilateral/square | שוה צלעות נצב זויות | term | ספר_ג'יבלי_אלמוקבאלא#DrKd | המרובע שוה הצלעות ונצב הזויות |
| quadrilateral/square | ר.ב.ע./מרובע | term | ספר_ג'יבלי_אלמוקבאלא#j1oW | מרובע |
| quadrilateral/square | שוה צלעות נצב זויות | term | ספר_ג'יבלי_אלמוקבאלא#Jhqq | שטח אחד שוה הצלעות ונצב הזויות |
| quadrilateral/square | שוה צלעות נצב זויות | term | ספר_ג'יבלי_אלמוקבאלא#HdgB | מרובע אחד שוה הצלעות נצב הזויות |
| quadrilateral/square | ר.ב.ע./מרובע | term | ספר_האלזיברא#mzGp | מרבע |
| quadrilateral/square | ר.ב.ע./מרובע | term | ספר_ג'יבלי_אלמוקבאלא#bhc1 | מרובעי' |
| quadrilateral/square | ש.ו.ה./שוה צלעות | term | ספר_ג'יבלי_אלמוקבאלא#Eq9Q | מרובעי' השוי הצלעות |
| quadrilateral/square | שוה צלעות נצב זויות | term | ספר_ג'יבלי_אלמוקבאלא#va50 | שטח שוה הצלעות נצב הזויות |
| quadrilateral/square | ש.ו.ה./שוה צלעות | term | ספר_ג'יבלי_אלמוקבאלא#tWbu | מרובע אחד שוה הצלעות מד' זויות |
| quadrilateral/square | term | ספר_המספר_/_אברהם_אבן_עזרא#IbQn | מרובע {{#annot:term|316|tTbn}}ארכו{{#annotend:tTbn}} כ{{#annot:term|317|FXNE}}רחבו{{#annotend:FXNE}} | |
| quadrilateral/square | ר.ב.ע./שטח מרובע | term | חשבון_השטחים#VRcA | שטח מרובע עליו |
| quadrilateral/square | ר.ב.ע./מרובע | term | חשבון_השטחים#OcVT | מרובע |
| quadrilateral/square | ר.ב.ע./מרובע | definition | ספר_היסודות_לאקלידס#yeYh | The '''square''' is that which is both equilateral and right-angled. הנה מהן המרובע הוא השוה הצלעות נצב הזויות |
| quadrilateral/square | ר.ב.ע./מרובע | term | ספר_האלזיברא#D62w | מרבע |
| quadrilateral/square | שוה צלעות נצב זויות | term | ספר_ג'יבלי_אלמוקבאלא#vsui | שטחי' שוי הצלעות והזויות נצבות |
| quadrilateral/square | שוה צלעות נצב זויות | term | ספר_ג'יבלי_אלמוקבאלא#wYJE | מרובע נצב הזויות שוה הצלעות |
| quadrilateral/square | שוה צלעות נצב זויות | term | ספר_ג'יבלי_אלמוקבאלא#d9eX | מרובע אחד שוה הצלעות והזיות נצבות |
| quadrilateral/square | ר.ב.ע./שטח מרובע | term | תחבולות_המספר#Q5y3 | שטח מרובע עליו |
| quadrilateral/square | כ.ו.נ./נכון | term | קצת_מענייני_חכמת_המספר#MiTK | מרובע נכון |
| quadrilateral/square | ר.ב.ע./מרובע | term | תחבולות_המספר#QPs4 | מרובע עליו |
| quadrilateral/square | ר.ב.ע./רבוע | definition | ספר_יסודי_התבונה_ומגדל_האמונה#YjuR | the first category is that whose four sides are all equal to each other in their value and each of its four angles is a right angle - this is called a square. החלק הראשון הוא אשר ארבע צלעיו כלם שוים בערכם אחת אל אחת וכן זויות ד' כל אחת מהן זוית נצבה וזה נקרא רבוע |
| quadrilateral/square | ר.ב.ע./מרובע | term | תחבולות_המספר#PFLT | מרובע |
| quadrilateral/square | ר.ב.ע./מרובע | term | האריתמטיקה_של_ניקומכוס#d8ge | מרובע |
| line/straight line | י.ש.ר./ישר | definition | ספר_היסודות_לאקלידס#lFcp | The '''straight line''' is that which lies straightly by the arrangement of points on it one by one. והקו הישר הוא המוצב על נכוחות אי זה נקודות יהיו עליו קצתם אל קצתם |
| line/straight line | י.ש.ר./מיושר | definition | ספר_יסודי_התבונה_ומגדל_האמונה#RN7a | The straight line is the one that passes directly from the two points that are its ends. והקו המיושר הוא ההולך לנכח ב' הנקדות אשר הם תכליתו |
| line/straight line | י.ש.ר./ישר | term | ספר_האלזיברא#4AfE | קו ישר |
| line/straight line | י.ש.ר./ישר | term | תחבולות_המספר#yJtO | קו ישר |
| line/straight line | י.ש.ר./מיושר | term | ספר_יסודי_התבונה_ומגדל_האמונה#iRJO | קו מיושר |
| line/straight line | י.ש.ר./ישר | term | חשבון_השטחים#FjaR | קו ישר |
| line/straight line | י.ש.ר./ישר | term | ספר_האלזיברא#wtKL | ישר |
| geometrical shape/surface | גג | term | משנת_המדות#RwBa | גג |
| geometrical shape/surface | ר.ק.ע./רקיע | term | ספר_יסודי_התבונה_ומגדל_האמונה#cV12 | הרקיע גלמי |
| geometrical shape/surface | ש.ט.ח./שטח | term | ספר_מעשה_חושב#tBy4 | שטחים |
| geometrical shape/surface | פ.ר.ש./פרוש | definition | ספר_יסודי_התבונה_ומגדל_האמונה#vPk1 | The second is called surface [lit. spread], and it is called by this name, since it is spread on the body as if it apart from it, and this body has length and breadth alone. והשני נק' פרוש וקראו לו בשם הזה מפני שהוא פרוש על הגולם כאלו היה חוצה ממנו וזה הפרוש יש לו ארך ורחב בלבד |
| geometrical shape/surface | ש.ט.ח./שטח | definition | האריתמטיקה_של_ניקומכוס#QKfY | Likewise, one may say that what has only length and breadth is a surface, and the surface is that which has only length and breadth. וכמו כן יאמר מה שהיה לו אורך ורחב לבד הוא שטח והשטח הוא מה שהיה לו אורך ורחב לבד |
| geometrical shape/surface | ש.ט.ח./שטח | term | האריתמטיקה_של_ניקומכוס#Lfgr | שטח |
| geometrical shape/surface | פ.ר.ש./פרוש | term | ספר_יסודי_התבונה_ומגדל_האמונה#Uads | פרושים |
| geometrical shape/surface | פ.ר.ש./פרוש | term | ספר_יסודי_התבונה_ומגדל_האמונה#aIkf | פרוש |
| geometrical shape/surface | ש.ט.ח./שטח | term | ספר_האלזיברא#eJNd | שטח |
| geometrical shape/surface | ש.ט.ח./שטח | term | בר_נותן_טעם#5Pke | שטח |
| geometrical shape/surface | ש.ט.ח./שטח | definition | האריתמטיקה_של_ניקומכוס#B67O | The two-dimensional is the surface. ומה שהיה בעל שני המשכים הוא שטח |
| geometrical shape/surface | ש.ט.ח./שטח | term | ספר_מעשה_חושב#KV4R | שטח |
| geometrical shape/surface | ש.ט.ח./שטח | term | תחבולות_המספר#JkjG | שטח |
| geometrical shape/surface | ש.ט.ח./שטח | term | מלאכת_המספר#T5GN | שטח |
| geometrical shape/surface | solid/volume | term | ספר_הכללים_במספר#F8HS | משיחת שטח הבריכה |
| geometrical shape/surface | מ.ש.ח./משיחה | term | ספר_הכללים_במספר#hb4l | משיחת שטח הכדור |
| geometrical shape/surface | ש.ט.ח./שטח | definition | מלאכת_המספר#gJ6u | the surface is a quantity that has length and breadth without depth, whose limits are two lines. השטח הוא כמות בעל אורך ו{{#annot:term|317,1488|SLfo}}רוחב {{#annotend:SLfo}}בלתי עומק ש{{#annot:term|279,1887|tYDo}}תכליותיו{{#annotend:tYDo}} ב' קוים |
| geometrical shape/surface | ש.ט.ח./שטח | term | ספר_ג'יבלי_אלמוקבאלא#fIHT | שטחי' |
| geometrical shape/surface | ש.ט.ח./שטח | term | חשבון_השטחים#fxB7 | שטח |
| geometrical shape/surface | ש.ט.ח./שטח | term | ספר_המספר_/_אליהו_מזרחי#GMrP | שטח |
| geometrical shape/surface | ש.ט.ח./שטח | term | ספר_המספר_/_אליהו_מזרחי#P6xS | שטחים |
| geometrical shape/surface | ש.ט.ח./שטח | definition | ספר_היסודות_לאקלידס#PhWl | The '''surface''' is that which has length and breadth only. והשטח הוא אשר לו אורך ורוחב לבד |
| geometrical shape/surface | ש.ט.ח./שטח | term | ספר_ג'יבלי_אלמוקבאלא#qKhs | שטח |
| geometrical shape/surface | גג | definition | משנת_המדות#KsSG | The surface itself is the area. והגג עצמו היא המשיחה |
| geometric relations/to be the side of an area | ח.ז.ק./הוחזק | term | ספר_ג'יבלי_אלמוקבאלא#i2ia | המוחזקי' ב |
| geometric relations/to be the side of an area | ח.ז.ק./החזיק | term | ספר_האלזיברא#3DEC | מחזקת ב |
| geometrical shape/to construct | ע.מ.ד./העמיד | term | ספר_היסודות_לאקלידס#TWKo | נעמיד על |
| geometrical shape/to construct | ע.ש.ה./עשה | term | קצור_המספר#FaQ9 | נעשה |
| geometrical shape/to construct | term | ספר_האלזיברא#Zpze | נעשה מן א"ב מרבע | |
| geometrical shape/to construct | term | ספר_האלזיברא#Mz5i | נעשה מן קו א"ג מרבע | |
| geometrical shape/to construct | term | תחבולות_המספר#GRES | נעשה על | |
| geometrical shape/to construct | term | תחבולות_המספר#48WG | עשה על | |
| geometrical shape/to construct | term | תחבולות_המספר#8stU | תשים על | |
| geometrical shape/to construct | term | תחבולות_המספר#yUj4 | נשים עליו | |
| geometrical shape/to construct | term | תחבולות_המספר#nGtX | נשים על | |
| geometrical shape/to construct | נ.ו.ח./הניח | term | חשבון_השטחים#cuCt | נניח על |
| geometrical shape/to construct | ע.ש.ה./עשה | term | חשבון_השטחים#ZiEA | נעשה על |
| geometrical shape/to construct | ע.ש.ה./עשה | term | חשבון_השטחים#6VxT | עשה על |
| geometrical shape/to construct | term | חשבון_השטחים#WKQn | נשים על | |
| geometrical shape/to construct | term | חשבון_השטחים#yth4 | אשים על | |
| geometrical shape/to construct | ע.מ.ד./העמיד | term | ספר_היסודות_לאקלידס#yirg | נעמיד על |
| line/to cut a line | ח.ל.ק./נחלק לחצאים | to be halved at point | תחבולות_המספר#JUPf | נחלק לחצאין על נקודת |
| line/to cut a line | ח.ל.ק./חלק קו | term | חשבון_השטחים#vclu | נחלק קו |
| line/to cut a line | ח.ל.ק./חלק קו | term | תחבולות_המספר#q7GH | נחלק קו |
| line/to cut a line | ח.ל.ק./נחלק לחצאים | to be halved at point | חשבון_השטחים#JnrH | נחלק לחצאים על נקודת |
| line/to cut a line | to be cut randomly at point | ספר_האלזיברא#S8Q5 | יחולק איך שקרה על נקדת | |
| line/to cut a line | to be halved at point | ספר_האלזיברא#Wk92 | נחלק לחצאין על נקדת | |
| line/to cut a line | ח.ל.ק./חלק לחצאים | to halve a line at point | חשבון_השטחים#g8x0 | תחלק קו א"ב לשני חצאים על |
| line/to cut a line | ח.ל.ק./חלק לחצאים | to halve a line at point | תחבולות_המספר#uwUl | נחלקהו לחציין על נקודת |
| line/to cut a line | ח.ל.ק./חלק לחצאים | to halve a line at point | תחבולות_המספר#vadk | נחלק אותו לחצאין על נקודת |
| line/to cut a line | ח.ל.ק./חלק לחצאים | to halve a line at point | חשבון_השטחים#l2ux | נחלק אותו לחציים על נקודת |
| line/to cut a line | to be cut into two unequal segments | חשבון_השטחים#Cbhd | נחלק לשני חלקים בלתי שוים על נקודת | |
| line/to cut a line | to be cut into two unequal segments | תחבולות_המספר#sLnn | נחלק לשני חלקים בלתי שוים על נקודת | |
| line/to cut a line | ח.ל.ק./נחלק | to be cut into two segments | תחבולות_המספר#ms8e | יחלק לשני חלקים |
| line/to cut a line | to cut randomly at point | ספר_האלזיברא#6A8l | חלק איך שקרה על נקדת | |
| line/to cut a line | ח.ל.ק./חלק קו | term | תחבולות_המספר#QaGp | תחלק קו |
| line/to cut a line | to halve a line | ספר_האלזיברא#CWXy | נחלקהו 130rלחצאין על נקדת | |
| line/to cut a line | ח.ל.ק./נחלק לחצאים | to be halved at point | חשבון_השטחים#Em05 | נחלק לחציים על נקודת |
| line/to cut a line | ח.ל.ק./חלק קו | term | חשבון_השטחים#zVhW | תחלק קו |
| line/to cut a line | to halve a line | ספר_האלזיברא#PkWc | נחלקהו לב' חלקי' שוים על נקדת | |
| line/to cut a line | to cut into two unequal segments | ספר_האלזיברא#fs2S | נחלקה לשני חלקים בלתי שוים על נקדת | |
| line/to cut a line | ח.צ.י./חצה | to halve a line at point | ספר_ג'יבלי_אלמוקבאלא#yo7t | נחצה לשני חלקים שוים בנקודת |
| geometric relations/to cut off | ב.ד.ל./הבדיל | term | תחבולות_המספר#ogvR | הבדלנו |
| geometric relations/to cut off | ב.ד.ל./הבדיל | term | תחבולות_המספר#Ptjj | נבדיל |
| geometric relations/to cut off | ב.ד.ל./הבדיל | term | חשבון_השטחים#ueVd | נבדיל |
| geometric relations/to cut off | ב.ד.ל./הבדיל | term | חשבון_השטחים#LmNg | תבדיל |
| geometric relations/to divide a figure | פ.ס.ק./פסק | term | משנת_המדות#rWbW | פסקה לשנים |
| geometric relations/to divide a figure | פ.ס.ק./פסק | term | משנת_המדות#pgwr | פוסקה שנים |
| geometry/to draw | י.צ.א./הוציא | to draw a line | תחבולות_המספר#vgQz | נוציא קוי |
| geometry/to draw | י.צ.א./הוציא | to draw a line | ספר_ג'יבלי_אלמוקבאלא#cMHc | תוציא קו אחד מ |
| geometry/to draw | י.צ.א./הוציא | term | תחבולות_המספר#eY6o | נוציא |
| geometry/to draw | י.צ.א./הוציא | term | חשבון_השטחים#2Qhs | תוציא מ |
| geometry/to draw | י.צ.א./הוציא | term | חשבון_השטחים#xLty | תוציא מנקודות |
| geometry/to draw | י.צ.א./הוציא | term | חשבון_השטחים#2Ih7 | תוציא מנקדות |
| geometry/to draw | י.צ.א./הוציא | term | חשבון_השטחים#3P9m | תוציא מנקודת |
| geometry/to draw | י.צ.א./הוציא | to draw a line | תחבולות_המספר#E2dh | תוציא קו |
| geometry/to draw | י.צ.א./הוציא | to draw a line | ספר_ג'יבלי_אלמוקבאלא#Z0HS | נוציא קו אחר ישר |
| geometry/to draw | י.צ.א./הוציא | to draw a line | חשבון_השטחים#DwRl | תוציא קו |
| geometry/to draw | צ.י.ר./צויר | term | ספר_ג'יבלי_אלמוקבאלא#9Ak5 | מצוייר |
| geometry/to draw | ק.ו.י./קוה | term | ספר_היסודות_לאקלידס#qfrQ | נקוה |
| geometry/to draw | ק.ו.י./קוה | term | ספר_היסודות_לאקלידס#kY7l | נקוה |
| geometry/to draw | ע.ש.ה./עשה | to draw a line | חשבון_השטחים#cXQo | לעשות קוי |
| geometry/to draw | ע.ב.ר./העביר | to draw a line | ספר_היסודות_לאקלידס#ztOj | נעביר קו |
| geometry/to draw | י.צ.א./הוציא | term | ספר_האלזיברא#DpZV | אוציא מ |
| geometry/to draw | ע.ב.ר./העביר | to draw a line | ספר_האלזיברא#Ii1B | נעביר קו |
| geometry/to draw | צ.י.ר./צייר | term | חשבון_השטחים#H57u | תצייר |
| geometry/to draw | term | ספר_האלזיברא#uW6o | צירתי | |
| geometry/to draw | י.צ.א./הוציא | to draw a line | מלאכת_המספר#DyfV | נוציא |
| geometry/to draw | צ.י.ר./צייר | term | ספר_ג'יבלי_אלמוקבאלא#MU8F | נצייר |
| geometry/to draw | י.צ.א./הוציא | to draw a line | מלאכת_המספר#utEh | נוציא |
| geometry/to draw | נ.ג.ע./הגיע | to draw a line | ספר_ג'יבלי_אלמוקבאלא#omwB | תגיע קו אחד מ |
| geometry/to draw | י.צ.א./הוציא | to draw a line | ספר_ג'יבלי_אלמוקבאלא#yF7x | נוציא קו |
| circle/to draw a circle | נ.ק.פ./הקיף | term | ספר_היסודות_לאקלידס#Wj8n | נקיף על |
| circle/to draw a circle | ח.ק.ק./לחוק | term | ספר_הכללים_במספר#CyVl | לחוק בעגול |
| circle/to draw a circle | נ.ק.פ./הקיף | term | ספר_היסודות_לאקלידס#Bgcx | נקיף עגולה |
| circle/to draw a circle | ע.ג.ל./עגל | term | ספר_יסודי_התבונה_ומגדל_האמונה#fYqf | לעגל עליו עגולה |
| circle/to draw a circle | ק.ו.י./קוה | term | ספר_היסודות_לאקלידס#Pgvr | נקוה עגולה |
| circle/to draw a circle | ח.ק.ק./לחוק | term | ספר_הכללים_במספר#Wkts | לחוק בה עגול |
| circle/to draw a circle | ע.ג.ל./עגל | term | ספר_יסודי_התבונה_ומגדל_האמונה#Oerg | לעגל בתוכו עגולה |
| circle/to draw a circle | ק.ו.י./קוה | term | ספר_היסודות_לאקלידס#MoiN | נקוה |
| geometric relations/to encompass | נ.ק.פ./הקיף | term | תחבולות_המספר#jij2 | יקיפו בו |
| geometric relations/to encompass | נ.ק.פ./הקיף | term | תחבולות_המספר#fbeH | מקיף |
| geometric relations/to encompass | נ.ק.פ./הקיף | term | ספר_מעשה_חושב#u8IF | המקיפים בו |
| geometric relations/to encompass | נ.ק.פ./הקיף | term | מלאכת_המספר#9qMC | מקיף אותו |
| line/to extend a line | מ.ש.כ./המשיך קו | term | חשבון_השטחים#Hlm5 | נמשיך קו |
| line/to extend a line | מ.ש.כ./המשיך קו | term | ספר_האלזיברא#67xx | נמשיך קו |
| line/to extend a line | מ.ש.כ./המשיך קו | term | תחבולות_המספר#KMfZ | נמשיך קו |
| line/to extend a line | מ.ש.כ./המשיך קו | term | ספר_ג'יבלי_אלמוקבאלא#zKyQ | תמשיך קו אחד עליו |
| surface/to form an area | term | תחבולות_המספר#RRCV | נשלים שטח | |
| surface/to form an area | ש.ל.מ./השלים | term | חשבון_השטחים#y3cp | נשלים שטח |
| geometric relations/to intersect | ח.ב.ר./מחובר | term | ספר_דיני_ממונות#tuwl | קוים מחוברים |
| geometric relations/to intersect | term | ספר_האלזיברא#UM04 | יפגוש קו | |
| geometric relations/to intersect | פ.ס.ק./הפסיק | term | משנת_המדות#MXl7 | מפסיקין זה את זה |
| geometric relations/to intersect | ח.ת.כ./חתך | term | ספר_האלזיברא#NwpI | יחתכו |
| geometric relations/to join | ש.ת.פ./שתף | term | תחבולות_המספר#LFhk | נשתף עמו |
| geometric relations/to join | ד.ב.ק./הדביק | term | תחבולות_המספר#iJG4 | נדביק |
| geometric relations/to join | ד.ב.ק./הדביק | term | ספר_ג'יבלי_אלמוקבאלא#yNEQ | נדביק |
| geometric relations/to join | ד.ב.ק./הדביק | term | ספר_ג'יבלי_אלמוקבאלא#8VeD | נדביק |
| geometric relations/to revolve around | ס.ב.ב./סבב | term | ספר_יסודי_התבונה_ומגדל_האמונה#Py8c | סובב עליו |
| geometric relations/to revolve around | ס.ב.ב./סבב | term | ספר_המספר_/_אברהם_אבן_עזרא#gOMD | סובבות את |
| geometric relations/to touch | מ.ש.ש./משש | term | ספר_יסודי_התבונה_ומגדל_האמונה#OH1T | מושש ל |
| geometric relations/to touch | מ.ש.ש./משש | term | ספר_יסודי_התבונה_ומגדל_האמונה#IbMX | מושש |
| quadrilateral/trapezoid | נ.ט.י./נוטה | definition | ספר_היסודות_לאקלידס#JU9N | The quadrilaterals that are other than the above-mentioned figures are called '''trapezia'''. ומה שהיה על זולת מה שספרנו מן התמונות בעלות ארבע צלעות תקרא הנוטה |
| quadrilateral/trapezoid | ק.ט.מ./קטומה | term | ספר_הכללים_במספר#4hrz | קטומת הראש |
| quadrilateral/trapezoid | ק.ט.מ./קטומה | term | ספר_הכללים_במספר#0OmP | קטומה |
| surface/triangle | ש.ל.ש./משולש | definition | ספר_יסודי_התבונה_ומגדל_האמונה#Trd6 | the first of them is the triangle which is the surrounded by three straight lines. הראשון מהם הוא המשלש והוא המקיף אותו ג' קוים ישרים |
| surface/triangle | ש.ל.ש./משולשת | term | משנת_המדות#rrKK | משלשת |
| surface/triangle | ש.ל.ש./משולש | term | מלאכת_המספר#kEE3 | משולש |
| surface/triangle | ש.ל.ש./משולש | definition | ספר_יסודי_התבונה_ומגדל_האמונה#rvtN | Explanation of the properties of the surface that has three sides called triangle !style="text-align:right;"|פירוש עניני השטח אשר לו שלשה צלעים ונקרא משלש |
| surface/triangle | term | ספר_המספר_/_אברהם_אבן_עזרא#VH1b | משולש | |
| surface/triangle | ש.ל.ש./משולש | term | ספר_יסודי_התבונה_ומגדל_האמונה#Sluz | משלש |
| surface/triangle | ש.ל.ש./משולש | term | ספר_יסודי_התבונה_ומגדל_האמונה#jMAM | משלשים |
| solid/truncated solid | ח.ס.ר./מחוסר | term | האריתמטיקה_של_ניקומכוס#EfLj | מחוסר |
| solid/truncated solid | ק.ט.ע./קטוע | term | משנת_המדות#njDm | קטוע |
| solid/truncated solid | ח.ס.ר./מחוסר | term | האריתמטיקה_של_ניקומכוס#fXNB | הגשם המחוסר |
| line/uniform line | פ.ר.ד./מתפרד | term | ספר_יסודי_התבונה_ומגדל_האמונה#Tv8Y | מתפרד |
| line/uniform line | פ.ר.ד./מתפרד | term | ספר_יסודי_התבונה_ומגדל_האמונה#Pmls | הקו המתפרד |
| geometry/upper base | פה | term | מלאכת_המספר#Nkoh | פיו |
| circle/versine | ח.צ.צ./חץ | definition | משנת_המדות#UTQn | The sagitta is the drawn from the middle of the arc until the middle of the chord, as it is said: ''they set their arrow on the bowstring'' [Psalms 11, 2]. והחץ הוא המשוך מאמצע הקשת לאמצע היתר שנ' ''כוננו חצם על יתר''תהילים יא, ב |
| circle/versine | term | ספר_המספר_/_אברהם_אבן_עזרא#WiTP | חץ | |
| circle/versine | ח.צ.צ./חץ | term | משנת_המדות#nlBR | חץ |
| solid/volume | מ.ש.ח./משיחה | term | משנת_המדות#g4GG | משיחת הגג |
| solid/volume | ש.ב.ר./תשבורת | term | ספר_הכללים_במספר#SpiW | תשבורת רבוע ה |
| solid/volume | ש.ב.ר./תשבורת | term | ספר_הכללים_במספר#RKIL | תשבורת גוף ה |
| solid/volume | ש.ב.ר./תשבורת | term | ספר_המספר_/_אליהו_מזרחי#0BrL | תשבורת |
| solid/volume | ש.ב.ר./תשבורת | term | ספר_הכללים_במספר#Lrs9 | תשבורת |
| solid/volume | מ.ש.ח./משיחה | term | משנת_המדות#z83J | משיחת הגוף |
| linear dimension/width | ר.ח.ב./רוחב | term | מלאכת_המספר#SLfo | רוחב |
| linear dimension/width | ר.ח.ב./רוחב | term | ספר_ג'יבלי_אלמוקבאלא#gKan | רוחב ה |
| linear dimension/width | ר.ח.ב./רוחב | term | קצור_המספר#Tl4A | רחבה |
| linear dimension/width | ר.ח.ב./רוחב | term | ספר_ג'יבלי_אלמוקבאלא#ytPj | רחב ה |
| linear dimension/width | ר.ח.ב./מרחב | term | ספר_ג'יבלי_אלמוקבאלא#nsj0 | מרחבם |
| linear dimension/width | ר.ח.ב./רוחב | term | ספר_ג'יבלי_אלמוקבאלא#P8YW | רחבו |
| linear dimension/width | ר.ח.ב./מרחב | term | ספר_ג'יבלי_אלמוקבאלא#4CJI | מרחב ה |
| linear dimension/width | ר.ח.ב./מרחב | term | ספר_ג'יבלי_אלמוקבאלא#jOGZ | מרחב |
| linear dimension/width | ר.ח.ב./רוחב | term | משנת_המדות#PREy | רחב |
| linear dimension/width | ר.ח.ב./רוחב | term | בר_נותן_טעם#Ykal | רוחב |
| linear dimension/width | ר.ח.ב./רוחב | term | מלאכת_המספר#DQWq | רוחב |
| linear dimension/width | term | ספר_המספר_/_אברהם_אבן_עזרא#FXNE | רחבו |
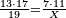
א.ו.ת.
[Collapse]אות: numeral
| Category | Category | Comment | Link | Annotated text | author | time |
|---|---|---|---|---|---|---|
| decimal positional system/numeral | א.ו.ת./אות | term | ספר_המספר_/_אליהו_מזרחי#EYyI | אות | Elijah Mizraḥi | 1450-1526 |
| decimal positional system/numeral | א.ו.ת./אות | term | ספר_אגריס#CMl9 | אותיות | 1300-1400 | |
| decimal positional system/numeral | א.ו.ת./אות | term | אגרת_המספר#BaEX | אותיות | Ibn al-Bannāʼ al-Marrākushī | 1350-1429/1433 |
| decimal positional system/numeral | א.ו.ת./אות | term | ספר_ציפרא#b7Xx | אותיו' | 1400-1500 | |
| decimal positional system/numeral | א.ו.ת./אות | term | אגרת_המספר#nckU | אות | Ibn al-Bannāʼ al-Marrākushī | 1350-1429/1433 |
| decimal positional system/numeral | א.ו.ת./אות | term | ספר_אגריס#vv7v | אות | 1300-1400 | |
| decimal positional system/numeral | א.ו.ת./אות | term | ספר_החשבון_לאל_חצאר#6oja | אות | Abū Bakr al-Haṣṣār | 1271 |
| decimal positional system/numeral | א.ו.ת./אות | term | בר_נותן_טעם#PBaR | אותיות | Jacob Canpanṭon | 1430 |
| decimal positional system/numeral | א.ו.ת./אות | term | קצור_המספר#Rmee | אותיות | Judah Ibn Verga | unknown-1450 |
| decimal positional system/numeral | א.ו.ת./אות | term | בר_נותן_טעם#EozN | אות | Jacob Canpanṭon | 1430 |
| decimal positional system/numeral | א.ו.ת./אות | term | קצור_המספר#mTHy | אות | Judah Ibn Verga | unknown-1450 |
| decimal positional system/numeral | א.ו.ת./אות | term | ספר_עיון_העקרים_לחשבון_ההנדיים#mq5P | אותיות | Kūšyār Ibn Labbān | 1450-1460 |
| decimal positional system/numeral | א.ו.ת./אות | term | עיר_סיחון#phuN | אותיות | Joseph Ben Moses Ṣarfati | unknown-1384 |
| decimal positional system/numeral | א.ו.ת./אות | term | ספר_עיון_העקרים_לחשבון_ההנדיים#9bnm | אות | Kūšyār Ibn Labbān | 1450-1460 |
| decimal positional system/numeral | א.ו.ת./אות | term | מלאכת_המספר#Duvu | אות | Isaac Ben Moses ‘Eli | 1400-1500 |
| decimal positional system/numeral | א.ו.ת./אות | term | ספר_החשבון_לאל_חצאר#yuS2 | אותיות | Abū Bakr al-Haṣṣār | 1271 |
| decimal positional system/numeral | א.ו.ת./אות | term | ספר_החשבון_לאל_חצאר#voac | אותיות | Abū Bakr al-Haṣṣār | 1271 |
| decimal positional system/numeral | א.ו.ת./אות | term | עיר_סיחון#OGlo | אות | Joseph Ben Moses Ṣarfati | unknown-1384 |
| Category | Comment | Link | Annotated text |
|---|---|---|---|
| word problem/find a number | a/b=¾;(ab)·(a+b)=a² | ספר_ג'יבלי_אלמוקבאלא#bWjS | Find me two numbers such that the one is a part of the other as 3 is of 4;
:and when the first is multiplied by the second, and the product is multiplied by the sum of both numbers together, it yields the same as the product of the larger by itself.
:I ask: how much will each number be?
: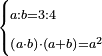 תמצא לי שני מספרים שיהיה חלק האחד מהאחר כמו שהוא ג' מד'
תמצא לי שני מספרים שיהיה חלק האחד מהאחר כמו שהוא ג' מד'וכאשר הוכה הראשון בשני ואותה ההכאה תוכה על מקובץ שני המספרי' יחד יעשה כמו הכאת הגדול בעצמו אשאל כמה יבא להיות כל אחד מהמספרי' |
| word problem/find a number | a/b=¾;a√8+b²=48 | ספר_ג'יבלי_אלמוקבאלא#aGAJ | Find me two numbers such that the one is a part of the other as 3 is of 4;
:and if the first is multiplied by the root of 8, and the second is multiplied by itself, then both products are summed together, it yields 48.
:I ask: how much will each number be?
: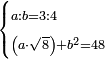 תמצא לי שני מספרי' שהאחד יהיה חלק מהאחר כמו שג' הוא מד'
תמצא לי שני מספרי' שהאחד יהיה חלק מהאחר כמו שג' הוא מד'ומוכה הראשון בשרש ח' והשני בעצמו ואותן שתי הכאות יחוברו יחד יעשו מ"ח אשאל כמה יבא להיות כל האחד מהמספרי' |
| word problem/find a number | 2/3=4/a | ספר_דיני_ממונות#PZIU | 248) Question: we have four numbers, such that the ratio of the first to the second is the same as the ratio of the third to the fourth, the sum of the four squares is 65, the first is 2, the second is 3, and the third is 4.
:I ask: how much is the fourth?
רמח) שאלה לנו ד' מספרים שיחס הא' אל הב' כיחס הג' אל הד' והד' מרובעים עולה ס"ה וממון הא' ב' וממון הב' ג' וממון הג' ד' אשאל כמה ממון הד' |
| word problem/find a number | a²·a=12a+10a² | ספר_ג'יבלי_אלמוקבאלא#FhTO | Find me a number, such that when it is multiplied by itself, and the product is multiplied by that number, it yields the same as the product of that number by 12, and that number multiplied by itself, then this product is multiplied by ten and this is added to the product by 12.
:I ask: how much will the number be?
: עשה לי החשבון תמצא לי מספר אחד שכאשר הוכה בעצמו ואותה ההכאה תוכה במספר ההוא יעשה כמו הכאת המספר האמור בי"ב ואח"כ להכות המספר האמור בעצמו ואותה ההכאה תוכה בעשרה ולחבר עם ההכאה שנעשתה בי"ב
עשה לי החשבון תמצא לי מספר אחד שכאשר הוכה בעצמו ואותה ההכאה תוכה במספר ההוא יעשה כמו הכאת המספר האמור בי"ב ואח"כ להכות המספר האמור בעצמו ואותה ההכאה תוכה בעשרה ולחבר עם ההכאה שנעשתה בי"באשאל כמה יבא להיות המספר האמור |
| word problem/find a number | repeated subtraction | מלאכת_המספר#TEa8 | Question: if we subtract 12 from the double of any number, then we subtract another 12 from double the remainder also, and double the remainder is 12, how much is the original number?
:![\scriptstyle2\sdot\left[\left[2\sdot\left(2X-12\right)\right]-12\right]=12](/mediawiki/images/math/2/e/6/2e6830e9068e310a2b310a61c4dc1e73.png) שאילה אם מכפלו של אי זה מספר נקח י"ב ומכפל הנשאר נקח ג"כ י"ב אחרי' וכפל הנשאר יהיה י"ב כמה היה המספר הראשון
שאילה אם מכפלו של אי זה מספר נקח י"ב ומכפל הנשאר נקח ג"כ י"ב אחרי' וכפל הנשאר יהיה י"ב כמה היה המספר הראשון |
| word problem/find a number | a+½¼a+¾a+¼¼a=2 | Arithmetic_Textbook_by_R._Aharon_ben_Isaac#EJ6w | The third question: what is the unknown number that if you add to it one-half of its quarter, its three quarter and one-quarter of its quarter, the total is but two?
: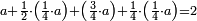 שאלה שלישית מהו המספר הנעלם אשר אם תחבר עליו חצי רביעיתו ושלושת רביעיתו ורביעית רביעיתו ולא יהיה אלא שנים
שאלה שלישית מהו המספר הנעלם אשר אם תחבר עליו חצי רביעיתו ושלושת רביעיתו ורביעית רביעיתו ולא יהיה אלא שנים |
| word problem/find a number | a+b=c;a+c=100b | מלאכת_המספר#Q70A | Question: if there are three numbers, the [sum of the] first and the second is as the third, and the [sum of the] first and the third is as a hundred of the second. How much is each of the three numbers? שאילה אם יהיו ג' מספרי' והראשון והשני יהיו בכמות השלישי והראשון והשלישי כמאה מן השני כמה יהיה כל אחד מהשלשה מספרי' |
| word problem/find a number | ¼a=⅓(a-¼a)=½((a-¼a)-⅓(a-¼a)) | ספר_דיני_ממונות#irk0 | Question: if you take any number, then you take its quarter from it, then a third of the remainder, then half the remainder after taking the third, all parts are the same. שאלהMS L שכ אם תחלק איזה מספר שיזדמן ותקח ממנו רביעיתו ומהנשאר שלישיתו ומהנשאר אחר לקיחת השליש מחציתו יש לכלם חלק שוה |
| word problem/find a number | √a+√(a-√a)=3 | ספר_דיני_ממונות#e6ph | 156) Question: if you want to find a number such that if you subtract its root and add it to the root of the remainder it makes 3.
: קנו)MS L: קנג שאלה אם תרצה למצא מספר אשר אם תוציא ממנו השרש ותחבר אותו עם השרש מהנשאר יעשה ג'
קנו)MS L: קנג שאלה אם תרצה למצא מספר אשר אם תוציא ממנו השרש ותחבר אותו עם השרש מהנשאר יעשה ג' |
| word problem/find a number | a/b=⅗;(a√a+b√b)√8=100 | ספר_ג'יבלי_אלמוקבאלא#dp2A | Find me two numbers such that the one is a part of the other as 3 is of 5;
:and when each is multiplied by its root and both products are summed together, then the sum is multiplied by the root of 8, it yields 100.
:I ask: how much will each number be?
:![\scriptstyle\begin{cases}\scriptstyle a:b=3:5\\\scriptstyle\left[\left(a\sdot\sqrt{a}\right)+\left(b\sdot\sqrt{b}\right)\right]\sdot\sqrt{8}=100\end{cases}](/mediawiki/images/math/7/1/1/71187e61ee927d7bba48ce232ff213cc.png) תמצא לי שני מספרי' שיהיה חלק האחד מהאחר כמו שהוא ג' מה'
תמצא לי שני מספרי' שיהיה חלק האחד מהאחר כמו שהוא ג' מה'וכשיוכה כל אחד על שרשו ויחוברו שתי אלו ההכאות יחד וזה הסך יוכה בשרש ח' יעשה מאה אשאל כמה יבא להיות כל אחד מהמספרים |
| word problem/find a number | a/b=⅔;a√a=2b | ספר_ג'יבלי_אלמוקבאלא#Jb23 | Find me two numbers such that the first is a part of the second as 2 is of 3;
:and when the first is multiplied by its root it yields the same as the product of the second by 2.
:I ask: how much will each number be?
: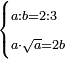 תמצא לי שני מספרים שיהיה הראשון חלק מהשני כמו שב' הוא מג'
תמצא לי שני מספרים שיהיה הראשון חלק מהשני כמו שב' הוא מג'וכשיוכה הראשון בשרש עצמו יעשה כמו הכאת השני בשנים אשאל כמה יבא להיות כל אחד מהמספרים |
| word problem/find a number | a/b=⅔;(ab)²=20+√30 | ספר_ג'יבלי_אלמוקבאלא#9iel | [Find me two numbers] such that one is a part of the other as 2 is of 3;
:and when the first is multiplied by the second and this product is multiplied by itself, it yields 20 and a root of 20.
:I ask: how much will each number be?
: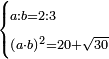 עשה לי זה החשבון שיהיה האחד חלק מהאחר כמו שב' הוא חלק מג'
עשה לי זה החשבון שיהיה האחד חלק מהאחר כמו שב' הוא חלק מג'ובהכות הראשון בשני ואותה ההכאה תוכה בעצמה יעשה כ' ושרש ל' אשאל כמה יבא להיות כל אחד מהמספרי' |
| word problem/find a number | a/b=¼;aba=³√216 | ספר_ג'יבלי_אלמוקבאלא#nkyB | Find me two numbers such that one is a part of the other as 1 is of 4;
:and the one multiplied by the other, and this product is multiplied by the first, yields a cube root of 216.
:I ask: how much will each number be?
:![\scriptstyle\begin{cases}\scriptstyle a:b=1:4\\\scriptstyle\left(a\sdot b\right)\sdot a=\sqrt[3]{216}\end{cases}](/mediawiki/images/math/1/7/1/171ce646462d735ef90bd02638d35efb.png) והנה המשל תמצא לי שני מספרי' שיהיה אחד מהם חלק מהאחר כמו שא' הוא מד'
והנה המשל תמצא לי שני מספרי' שיהיה אחד מהם חלק מהאחר כמו שא' הוא מד'ומוכה הראשון בשני וההכאה ההיא תוכה בראשון יעשה שרש מעו' מרי"ו אשאל כמה יבא להיות כל אחד מהמספרי' |
| word problem/find a number | six proportional numbers | ספר_דיני_ממונות#Wrir | For example: we have six proportional numbers, the first is 2 and the sixth is 64.
::We wish to know which is the second.
דמיון זה יש לנו ו' {{#annot:term|994,1277|VUeX}}מספרים מתיחסים{{#annotend:VUeX}} הראשון ב' והו' ס"ד נרצה לדעת מהו השני |
| word problem/find a number | a²=2b+8;a²+b²=80 | ספר_ג'יבלי_אלמוקבאלא#Ob3R | Find me two numbers such that the one multiplied by itself yields 2 times the second and 8 more;
:and when each of them is multiplied by itself, and the two products are summed together, they yield 80.
:I ask: how much will each number be?
: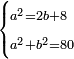 והנה המשל עשה לי זה החשבון תמצא לי ב' מספרי' שכאשר יוכה הראשון בעצמו יעשה ב' דמיוני השני וח' יותר
והנה המשל עשה לי זה החשבון תמצא לי ב' מספרי' שכאשר יוכה הראשון בעצמו יעשה ב' דמיוני השני וח' יותרומוכה כל אחד מהם בעצמו ויקובצו ב' ההכאו' יחד יעשו פ' אשאל כמה יבא להיות כל אחד מהמספרי' |
| word problem/find a number | (a-⅓a)²=20 | ספר_האלזיברא#xJ4O | Question: I want to find a number such that when its third is subtracted from it, the square of the remainder is 20.
: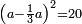 שאלה רציתי למצא מספר כאשר חסר ממנו שלישיתו מרבע הנשאר הוא מספר כ'
שאלה רציתי למצא מספר כאשר חסר ממנו שלישיתו מרבע הנשאר הוא מספר כ' |
| word problem/find a number | (a+b)/(c-a)=4½;(a+c)/(b-a)=5 | ספר_מעשה_חושב#UAnP | Example: the ratio of the first and the second summed together to what remains from the third when the first is subtracted from it is 4 integers and a half.
::The ratio of the first and the third summed together to what remains from the second when the first is subtracted from it is 5 integers.
::We wish to know: how much is each of the numbers that meet this condition?
:: והמשל שיהיה יחס הראשון והשני מקובצים אל הנשאר [מהשלישי כשחוסר ממנו הראשון ד' שלמים וחצי
והמשל שיהיה יחס הראשון והשני מקובצים אל הנשאר [מהשלישי כשחוסר ממנו הראשון ד' שלמים וחציויחס הראשון והשלישי מקובצים אל הנשאר] מהשני כשחוסר ממנו הראשון הוא ה' שלמים ונרצה לדעת כמה כל המספרים שינהגו זה המנהג |
| word problem/find a number | a/b=⅔;ab=20+√8 | ספר_ג'יבלי_אלמוקבאלא#B6TE | Find me two numbers such that the first is a part of the second as 2 is of 3;
:and when the first is multiplied by the second it yields 20 and a root of 8.
:I ask: how much will each number be?
: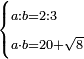 תמצא לי שני מספרים שיהיה הראשון חלק מהשני כמו שב' הוא מג'
תמצא לי שני מספרים שיהיה הראשון חלק מהשני כמו שב' הוא מג'ומוכה הראשון בשני יעשה כ' ושרש ח' אשאל כמה יבא להיות כל אחד מהמספרי' |
| word problem/find a number | a+⅓a+⅖a=81 | ספר_דיני_ממונות#khzV | 136) Question: find me a number such that if you add to it its third and its two fifths it makes [81].
: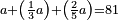 קלו)MS L: קלג שאלה תמצא לי מספר אשר אם תוסיף עליו שלישיתו ושני חמישיותיו יעשה י"ח
קלו)MS L: קלג שאלה תמצא לי מספר אשר אם תוסיף עליו שלישיתו ושני חמישיותיו יעשה י"ח |
| word problem/find a number | b-1=a+1;2(a-1)=b+1 | מלאכת_המספר#4BJp | Knowing the relation of two numbers that have the property that if we subtract one from the greater number and add it to the smaller number, the two numbers become equal; and if we subtract one from the smaller number and add it to the greater, the greater becomes double the smaller, or more if we wish ==== |style="width:45%; text-align:right;"|הפרק השני בידיעת התייחסות שני מספרי' שיש להם זה הטבע שאם נחסר אחד מהמספר הגדול ונוסיפהו על המספר הקטן יהיו הב' מספרי' שוים ואם נחסר אחד מהמספר הקטן ונוסיפהו בגדול יהיה הגדול כפל הקטן או יותר אם נרצה |
| word problem/find a number | a-(½a+½)-(½(a-(½a+½))+½)-(½(a-(½a+½)-(½(a-(½a+½))+½))+½) | קצור_המספר#9olE | As when one asks: what is the number such that when we subtract its half and one half more, then [we subtract] from the remainder its half and one half more, then [again we subtract] from the [second] remainder its half and one half more, we are left with one. כמו ששאל אחד איזהו מספר אשר כשנשליך חציו ועוד חצי אחד ומן הנשאר חציו ועוד חצי אחד וישאר אחד ב[ע]ינו |
| word problem/find a number | repeated subtraction | ספר_חשבון#QPoG | If one asks you: a number, you subtract a tenth from it, then you subtract again a tenth from the remainder, and 100 are left.
:How much was the original number before subtracting the two tenths? : ![\scriptstyle X-\frac{1}{10}X-\left[\frac{1}{10}\sdot\left(X-\frac{1}{10}X\right)\right]=100](/mediawiki/images/math/c/a/9/ca950635d00da924119bda8eef8b09bd.png) אם ישאלך אדם חשבון שהסרות ממנו הי' ומן הנשאר הסרות ממנו העשירית פעם שנית ונשארו ק'
אם ישאלך אדם חשבון שהסרות ממנו הי' ומן הנשאר הסרות ממנו העשירית פעם שנית ונשארו ק'כמה היה כל החשבון בטרם שהסרת ממנו ב' העשיריות |
| word problem/find a number | a+2⅔a=11 | Arithmetic_Textbook_by_R._Aharon_ben_Isaac#ZVW2 | First question: What is the number to which you add its two and 2-thirds and it is eleven?
: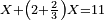 שאלה ראשונה מהו המספר 119r / [ק"א][אשר תחבר עליו שנים וב' שלישיות ויהיו] אחד עשר
שאלה ראשונה מהו המספר 119r / [ק"א][אשר תחבר עליו שנים וב' שלישיות ויהיו] אחד עשר |
| word problem/find a number | a/b=⅘;(ab)²=a²b | ספר_ג'יבלי_אלמוקבאלא#Ukgo | Find me two numbers such that the first is a part of the second as 4 is of 5;
:and when the first is multiplied by the second, and this product is multiplied by itself, it yields the same as the product of the first by itself, then that product is multiplied by the second number.
:I ask: how much will each number be?
: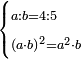 תמצא לי שני {{#annot: term | #proportional, #מתיחס | lK1I}}מספרים מתיחסים{{#annotend:lK1I}} שיהיה חלק הראשון מהשני כמו שהוא ד' מה'
תמצא לי שני {{#annot: term | #proportional, #מתיחס | lK1I}}מספרים מתיחסים{{#annotend:lK1I}} שיהיה חלק הראשון מהשני כמו שהוא ד' מה'וכשיוכה הראשון בשני ואותה ההכאה תוכה בעצמה ויעשה כמו שעושה הראשון מוכה בעצמו ואותה ההכאה תוכה במספר השני אשאל כמה יבא להיות כל אחד מהמספרים |
| word problem/find a number | a²=40+41\b | ספר_דיני_ממונות#aiR7 | 112) Question: find a number such that when you multiply it by itself the result is 40 and 41 parts of a fraction.
: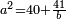 קיב)MS L: קי שאלה תמצא לי מספר אשר כשתכפלהו על עצמו יעלו מ' ומ"א חלקים מאיזה שבר שיזדמן
קיב)MS L: קי שאלה תמצא לי מספר אשר כשתכפלהו על עצמו יעלו מ' ומ"א חלקים מאיזה שבר שיזדמן |
| word problem/find a number | a(mod2)=1,a(mod3)=1,a(mod)4=1,a(mod5)=1,a(mod6)=1,a(mod7)=0 | ספר_המספר_/_אליהו_מזרחי#5uWz | Question: if one asks: what is the number that when counted by twos - one remains; by threes - one remains; by fours - one remains; by fives - one remains; by sixes - one remains; and by sevens - nothing remains. שאלה אם שאל שואל איזהו המספר אשר כשימנה מב' ב' ישאר אחד ומג' ג' ישאר אחד ומד' ד' ישאר אחד ומה' ה' ישאר אחד ומו' ו' ישאר אחד ומז' לא ישאר דבר |
| word problem/find a number | a+¼(b+c)=b+⅙(a+c)=c+⅑(a+b);b=20 | ספר_מעשה_חושב#sryH | Example: the first with a quarter of the rest is equal to the second with a sixth of the rest and is also equal to the third with a ninth of the rest.
::The first is added to the greatest part of the rest and the third is added to the smallest part of the rest.
::The second number is 20.
::We wish to know: how much is each of the rest?
::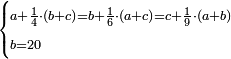 והמשל שיהיה הראשון עם רביעית הנשארים שוה אל השני עם ששית הנשארים
והמשל שיהיה הראשון עם רביעית הנשארים שוה אל השני עם ששית הנשאריםוהוא גם כן שוה אל השלישי עם תשיעית הנשארים וכבר התבאר לך במאמר הראשון מזה הספר שסדר אלו המספרים השלשה הוא על זאת ההדרגה אשר סדרנו רצוני שהראשון הוא אשר יתחבר עמו חלק יותר גדול מהנשארים והשלישי הוא אשר יתחבר עמו חלק יותר קטן מהנשארים והזכרתי לך זה למען לא תתבלבל בסדורם ויהיה המספר השני מהם כ' ורצינו לדעת כמה כל אחד מהנשארים |
| word problem/find a number | six proportional numbers | ספר_דיני_ממונות#FvyI | Example: the first is 2-[eighths] and the sixth is 256 integers. דמיון הראשון ב' שלישיות והו' רנ"ו שלמים |
| word problem/find a number | (a²·a)+b²=12½c | ספר_ג'יבלי_אלמוקבאלא#iH47 | Find me three numbers, such that the first is a part of the second as 2 is of 3, and the second is of the third as 3 is of 4.
:If the first is multiplied by itself, then the product is multiplied by the first number, and the second number is multiplied by itself, when both products are summed together, its yields the same as the product of the third number by 12 and a half.
: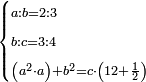 והנה המשל תמצא לי שלשה מספרים שיהיה חלק הראשון מהשני כמו שהוא ב' מג'
והנה המשל תמצא לי שלשה מספרים שיהיה חלק הראשון מהשני כמו שהוא ב' מג'והשני יהיה מהשלישי כמו שהוא ג' בד' ותכה הראשון בעצמו ואח"כ יוכה העולה במספר הראשון בעצמו ואח"כ יוכה המספר השני בעצמו וכשיחוברו יחד שתי אלו ההכאות יעשה כמו הכאת המספר השלישי בי"ב וחצי אשאל כמה יבא להיות כל אחד מהמספרים |
| word problem/find a number | a+½a+⅕a+⅐a=2 | Arithmetic_Textbook_by_R._Aharon_ben_Isaac#hxby | Question one: [What is] the number, such that if you add [to it] its half, its fifth, and its seventh, [the total is 2].
: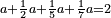 97r / ע"ט[...] [שאלה ראשונה] [מהו ה]מספר אשר אם תחבר [עליו] חציו חמשיתו ושביעיתו [יהיה עם הכל שנים]
97r / ע"ט[...] [שאלה ראשונה] [מהו ה]מספר אשר אם תחבר [עליו] חציו חמשיתו ושביעיתו [יהיה עם הכל שנים] |
| word problem/find a number | 3/a=5+7/b | ספר_דיני_ממונות#iyBa | 155) Question: if you want to find a number such that if you divide 3 by it, the result is 5 and 7 remain.
: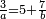 קנה)MS L: קנב שאלה אם תרצה למצא מספר אשר אם תחלק ג' מאותו המספר יבא ה' וישאר ז'
קנה)MS L: קנב שאלה אם תרצה למצא מספר אשר אם תחלק ג' מאותו המספר יבא ה' וישאר ז' |
| word problem/find a number | a+28=2a² | ספר_האלזיברא#AZ3B | Question: we want to find a number such that when we add to it 28 the sum is twice its square
: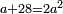 שאלה רצינו למצא מספר כאשר הוספנו עליו כ"ח {{#annot: term | #to be equal to, #שוה | hkKx}}יהיה שוה ל{{#annotend:hkKx}}שני דמיוני מרבעו
שאלה רצינו למצא מספר כאשר הוספנו עליו כ"ח {{#annot: term | #to be equal to, #שוה | hkKx}}יהיה שוה ל{{#annotend:hkKx}}שני דמיוני מרבעו |
| word problem/find a number | a/b=⅔;a√a=b² | ספר_ג'יבלי_אלמוקבאלא#LtCT | Find me two numbers such that one is a part of the other as 2 is of 3;
:and when the first is multiplied by its root it yields the same as the product of the second by itself.
:I ask: how much will each number be?
: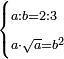 תמצא לי ב' מספרי' שיהיה האחד חלק מהאחר כמו ב' מג'
תמצא לי ב' מספרי' שיהיה האחד חלק מהאחר כמו ב' מג'ובהכות הראשו' בשרשו יעשה כמו הכאת השני בעצמו אשאל כמה יבא להיות כל אחד מן המספרים |
| word problem/find a number | b/(c-a)=3⅓;c/(b-a)=6 | ספר_מעשה_חושב#353o | Example: the ratio of the second to what remains from the third when the first is subtracted from it is 3 integers and a third.
::The ratio of the third to what remains from the second when the first is subtracted from it is the number 6.
::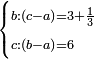 והמשל שיהיה יחס 82vהשני אל הנשאר מהשלישי כשחוסר ממנו הראשון ג' שלמים ושליש
והמשל שיהיה יחס 82vהשני אל הנשאר מהשלישי כשחוסר ממנו הראשון ג' שלמים ושלישויחס השלישי אל הנשאר מהשני כשחוסר ממנו הראשון מספר ו' |
| word problem/find a number | ⅓a+¼a=20 | מלאכת_המספר#Auzt | Example: we wish to find a number that the sum of its third and its quarter is 20.
: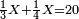 המשל רצינו למצא מספר שקבוץ שלישיתו ורביעיתו יהיה כ'
המשל רצינו למצא מספר שקבוץ שלישיתו ורביעיתו יהיה כ' |
| word problem/find a number | b=2a;a²b=9b | ספר_ג'יבלי_אלמוקבאלא#ZaKZ | Find me two numbers such that the one is double the other;
:and when the smaller is multiplied by itself, and the product is multiplied by the second number, it yields the same as the second, i.e. the larger, multiplied by 9.
:I ask: how much will each number be?
: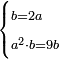 תמצא לי שני מספרים שהאחד יהיה {{#annot: term | #double, #כפל | HqHc}}כפל ה{{#annotend:HqHc}}שני
תמצא לי שני מספרים שהאחד יהיה {{#annot: term | #double, #כפל | HqHc}}כפל ה{{#annotend:HqHc}}שניוכשהוכה הקטון בעצמו ואותה ההכאה תוכה במספר השני יעשה כמו השני רצו' הגדול מוכה על ט' אשאל כמה יבא להיות כל אחד מהמספרים |
| word problem/find a number | a/b=¾;(ab)²+27=9b² | ספר_ג'יבלי_אלמוקבאלא#gmur | Find me two numbers such that the first is a part of the other as 3 is of 4;
:and the first multiplied by the second, and this product is multiplied by itself and 27 is added to it, yield the second number multiplied by itself and this product is multiplied by 9.
:I ask: how much will each number be?
: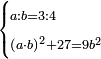 עשה לי זה החשבון תמצא לי שני מספרי' שיהיה הראשון חלק מהשני כמו שג' הוא מד'
עשה לי זה החשבון תמצא לי שני מספרי' שיהיה הראשון חלק מהשני כמו שג' הוא מד'ומוכה הראשון בשני ואותה ההכאה תוכה בעצמה ויחובר עמה כ"ז יעשה המספר השני בכפלו בעצמו ואותה ההכאה תוכה בט' אשאל כמה הוא כל אחד מהמספרים האמורי' |
| word problem/find a number | (a·⅔a)·a=a√8 | ספר_ג'יבלי_אלמוקבאלא#iQR6 | Find me a number, such that when it is multiplied by its two thirds, and this product is multiplied by that number, it yields the same as the product of that number by the root of 8.
:I ask: how much will the number be?
: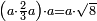 תמצא לי מספר אחד שכשיוכה בשני שלישיו ואותה ההכאה תוכה במספר האמור יעשה כמו הכאת המספר ההוא בשרש ח'
תמצא לי מספר אחד שכשיוכה בשני שלישיו ואותה ההכאה תוכה במספר האמור יעשה כמו הכאת המספר ההוא בשרש ח'אשאל כמה הוא כל אחד מהמספרי' |
| word problem/find a number | a(mod2)=1,a(mod3)=1,a(mod)4=1,a(mod5)=0 | ספר_המספר_/_אליהו_מזרחי#msZ5 | Likewise: if one asks: what is the number that when counted by twos - one remains; by threes - one remains; by fours - one remains; and by fives - nothing remains. וכן אם שאל שואל איזהו המספר אשר כשימנה מב' ב' ישאר אחד ומג' ג' ישאר אחד ומד' ד' ישאר אחד ומה' ה' לא ישאר דבר |
| word problem/find a number | a/b=⅔;ab√8=100 | ספר_ג'יבלי_אלמוקבאלא#mV9d | Find me two numbers such that one is a part of the other as 2 is of 3;
:and when the one is multiplied by the other, and the product is multiplied by the root of 8, it yields 100.
:I ask: how much will each number be?
: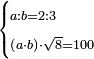 תמצא לי שני מספרי' שיהיה חלק האחד מהאחר כמו שב' הוא מג'
תמצא לי שני מספרי' שיהיה חלק האחד מהאחר כמו שב' הוא מג'וכשיוכה האחד באחר ואותה ההכאה תוכה בשרש ח' ועושה ק' אשאל כמה יבא להיות כל אחד מהמספרי' |
| word problem/find a number | a/b=⅘;ab=b√8 | ספר_ג'יבלי_אלמוקבאלא#roVS | Find me two numbers such that the first is a part of the second as 4 is of 5;
:and the one multiplied by the other yields the same as the product of the second by the root of 8.
:I ask: how much will each number be?
: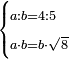 תמצא לי ב' מספרי' שיהיה הראשון חלק מהשני כמו שד' הוא מה'
תמצא לי ב' מספרי' שיהיה הראשון חלק מהשני כמו שד' הוא מה'ומוכה האחד באחר יעשה כמו הכאת השני בשרש ח' אשאל כמה יבא להיות כל אחד מהמספרי' |
| word problem/find a number | a²=4(a+8) | ספר_המספר_/_אליהו_מזרחי#OOxP | The example: if you want to know what is the number whose square equals the product of 4 by the sum of that number with 8.
::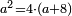 המשל בזה אם רצית לדעת איזהו המספר אשר יהיה מרובעו שוה לעולה מהכאת הד' עם המספר ההוא מחובר עם ח'
המשל בזה אם רצית לדעת איזהו המספר אשר יהיה מרובעו שוה לעולה מהכאת הד' עם המספר ההוא מחובר עם ח' |
| word problem/find a number | 4a(mod)6=1 | ספר_דיני_ממונות#alcr | Find me a number such that when I multiply it by 4, then divide it by 6, the remainder is 1.
: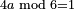 שאלה תמצא לי מספר שכשאכפלהו בד' ותחלקהו על ו' שישאר א'
שאלה תמצא לי מספר שכשאכפלהו בד' ותחלקהו על ו' שישאר א' |
| word problem/find a number | a/b=⅔;a²b+\sqrt{a²b}=342 | ספר_ג'יבלי_אלמוקבאלא#oR0N | Find me two numbers such that the first is a part of the second as 2 is of 3;
:and if the first is multiplied by itself, then this product is multiplied by the second, and this product is summed with its root, it yields 342.
:I ask: how much will each number be?
: עשה לי זה החשבון תמצא לי שני מספרי' שיהיה הראשון חלק מהשני כמו שב' הוא מג'
עשה לי זה החשבון תמצא לי שני מספרי' שיהיה הראשון חלק מהשני כמו שב' הוא מג'ומוכה הראשון בעצמו ואותה ההכאה תכה בשני ותחובר זאת ההכאה עם שרשה יעשה שמ"ב אשאל כמה יבא להיות כל אחד מהמספרי' |
| word problem/find a number | a/b=⅔;(ab)²+a²√4=4¼ | ספר_ג'יבלי_אלמוקבאלא#jPKB | Find me two numbers such that one is a part of the other as 2 is of 3;
:and when the first is multiplied by the second and this product is multiplied by itself then summed with the product of the first multiplied by itself then by the root of 4, it yields 4 and a quarter.
:I ask: how much will each number be?
: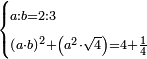 עשה לי זה החשבון תמצא לי שני מספרי' שיהיה הא' חלק מהאחר כמו שב' הוא מג'
עשה לי זה החשבון תמצא לי שני מספרי' שיהיה הא' חלק מהאחר כמו שב' הוא מג'ומוכה הראשון בשני וזאת ההכאה תוכה בעצמה ותחובר זאת ההכאה עם הכאת הראשון מוכה בעצמו ומה שיבא בשרש ד' יעשה ד' ורביע אשאל כמה יבא להיות כל אחד מהמספרי' |
| word problem/find a number | 37a=49 | Arithmetic_Textbook_by_R._Aharon_ben_Isaac#b6Ce | The [sixth] question: what is the number, such that when we multiply it by 37, the product is 49.
: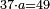 שאלה חמשית מהו המספר אשר כפלנו אותו על ל"ז והיוציא מן הכפל יהיה מ"ט
שאלה חמשית מהו המספר אשר כפלנו אותו על ל"ז והיוציא מן הכפל יהיה מ"ט |
| word problem/find a number | a²+19=b² | ספר_דיני_ממונות#DmeO | 149) Question: if you want to find a square number such that if you add to it 19 it will be a square.
: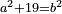 קמט)MS L: קמו שאלה אם תרצה למצא מספר מרובע אשר אם תשים עליו י"ט יהיה מרובע
קמט)MS L: קמו שאלה אם תרצה למצא מספר מרובע אשר אם תשים עליו י"ט יהיה מרובע |
| word problem/find a number | a/b=⅔;3a+4√b=30 | ספר_ג'יבלי_אלמוקבאלא#37bI | Find me two numbers such that the one is a part of the other as 2 is of 3;
:and when the first is multiplied by 3 and the root of the second by 4, then both the products are summed together, it yields thirty.
:I ask: how much will each number be?
: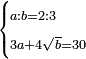 והנה המשל תמצא לי שני מספרי' שיהיה האחד חלק מאחר כמו שב' הוא מג'
והנה המשל תמצא לי שני מספרי' שיהיה האחד חלק מאחר כמו שב' הוא מג'ובהכות הראשון בג' ושרש השני בד' ויחוברו שתי אלו ההכאות יחד יעשה שלשים אשאל כמה יבא להיות כל אחד מהמספרי' |
| word problem/find a number | six proportional numbers | ספר_דיני_ממונות#fNcE | Example: we have six proportional numbers, the first of which is 2 and the sixth is 64. דמיון יש לנו ו' מספרים מתיחסים שהראשון ב' והו' ס"ד |
| word problem/find a number | six proportional numbers | ספר_דיני_ממונות#Yfpk | 111) Question: if you want to find a proportional number:
:For example: we have six proportional numbers, i.e. the ratio of the sixth to the fifth is the same as the ratio of the fifth to the fourth, and the same as the ratio of the fourth to the third, and the same as the ratio of the third to the second, and the same as the ratio of the second to the first.
:The first number is 2.
:The sixth is 2048.
:We wish to know how much is the second?
קיא)MS L: קט שאלה אם תרצה למצא {{#annot:term|994,2263|2xtB}}מספר יחסיי{{#annotend:2xtB}} הנקרא בלשונם {{#annot:term|994|DhLM}}נומירו פרופורציאונלי{{#annotend:DhLM}} דמיון זה יש לנו ו' {{#annot:term|994,2263|jjwV}}מספרים יחסיים{{#annotend:jjwV}} ר"ל שיחס הו' אל הה' כיחס הה' אל הד' וכיחס הד' אל הג' וכיחס הג' אל הב' וכיחס הב' אל הא' והמספר הא' ב' והו' ב' אלפים ומ"ח ונרצה לדעת מהו השני |
| word problem/find a number | (a+b)\c=3⅖⅐;(a+c)\b=7⅔¼;b=30 | ספר_דיני_ממונות#OJ9R | 58) Question: when one number is added to a second number, their ratio to the third is 3 integers, 2-fifths and a seventh.
:When the first is added to the third, their ratio to the [second] is 7 integers, 2-thirds and a quarter.
:The second number is 30.
:We wish to know: how much is each of the rest?
נח) שאלה שיהיה מספר ראשון כשחובר אל מספר שני היה יחסו אל הג' ג' שלמים וב' חמישיות ושביעיות וכשחובר הראשון אל הג' היה יחסו הראשון ז' שלמים וב' שלישיות ורביעיות והמספר הב' ל' רצינו לדעת כמה כל א' מהנשארים |
| word problem/find a number | perfect number | ספר_דיני_ממונות#x3Is | 109) If you want to find the second [perfect number] קט)MS L: om. ואם תרצה למצא השני |
| word problem/find a number | repeated subtraction | מלאכת_המספר#j27H | Question: if the half of any amount plus one are subtracted, then the half of the remainder plus one, then the half of the remainder plus one, and one remains, how much is the original number?
שאילה אם מאי זה כמות ילקח חציו ואחד יותר ומהנשאר חציו ויותר אחד ומהנשאר חציו ויותר אחד ונשאר אחד כמה היה המספר הראשון
|}
:![\scriptstyle\left[\left[X-\left(\frac{1}{2}X+1\right)\right]-\left[\left[\frac{1}{2}\sdot\left[X-\left(\frac{1}{2}X+1\right)\right]\right]+1\right]\right]-\left[\left[\frac{1}{2}\sdot\left[\left[X-\left(\frac{1}{2}X+1\right)\right]-\left[\left[\frac{1}{2}\sdot\left[X-\left(\frac{1}{2}X+1\right)\right]\right]+1\right]\right]\right]+1\right]=1](/mediawiki/images/math/b/5/0/b50e38940b92660b687bec29a547c334.png) |
| word problem/find a number | a/b=⅗;(ab)²=a² | ספר_ג'יבלי_אלמוקבאלא#0dhl | Find me two numbers such that the first is a part of the second as 3 is of 5;
:and when the smaller is multiplied by the greater and the product is multiplied by itself, it yields the same as the product of the greater by itself.
:I ask: how much will each number be?
: תמצא לי שני מספרים שיהיה חלק הראשון מהשני כמו שהוא ג' מה'
תמצא לי שני מספרים שיהיה חלק הראשון מהשני כמו שהוא ג' מה'וכאשר הוכה הקטון בגדול ומה שיעלה יוכה בעצמו יעשה כמו הכאת הגדול בעצמו אשאל כמה כל אחד מהמספרים |
| word problem/find a number | (a+b)/(c-a)=4½;(a+c)/(b-a)=5 | ספר_דיני_ממונות#UcvZ | 61) Question: the ratio of the first and the second summed together to what remains from the third when the first is subtracted from it is 4 integers and a half.
:The ratio of the first and the third summed together to what remains from the second when the first is subtracted from it is 5 integers.
:We wish to know: how much is each of the numbers that meet this condition?
סא) שאלה שיהיה יחס הראשון והשני מקובצים אל הנשאר מהג' כשחוסר ממנו הראשון ד' שלמים וחצי ויחס הראשון והג' מקובצים אל הנשאר מהב' כשחוסר ממנו הראשון הוא ה' שלמים ונרצה לדעת כמה כל המספרים שינהגו זה המנהג |
| word problem/find a number | seven proportional numbers | ספר_דיני_ממונות#nCKV | 258) Question of seven numbers: the first is 1 and the last is 729. רנח) שאלה מז' מספרים הראשון א' והאחרון תשכ"ט |
| word problem/find a number | 13·7·3a=3 | Arithmetic_Textbook_by_R._Aharon_ben_Isaac#o8DN | The eighth question: what is the number, such that when we multiply it by 3, then the product by 7, then the product by 13, the result is only 3.
: שאלה שמינית מהוא המספר אשר כפלנו אותו על ג' והיוציא על ז' והיוציא על י"ג ושלא יעלה אלא ג'
שאלה שמינית מהוא המספר אשר כפלנו אותו על ג' והיוציא על ז' והיוציא על י"ג ושלא יעלה אלא ג' |
| word problem/find a number | perfect number | ספר_דיני_ממונות#IXt8 | 108) Question: if you want to find a perfect number קח) שאלה אם תרצה למצא {{#annot:term|75|xt6c}}מספר שלם{{#annotend:xt6c}} נקרא בלשונם {{#annot:term|75|aiWm}}נומירו פירפיטו{{#annotend:aiWm}} |
| word problem/find a number | a·b·c=1000;a\b\c | ספר_דיני_ממונות#WgAN | 452) Question: we have three proportional numbers such that the ratio of the first to the second is as the ratio of the second to the third.
:If we multiply the first by the second, then multiply the result by the third it will be a thousand.
:How much are the proportional numbers?
116rתנב) שאלה יש לנו ג' מספרים יחסיים אשר יחס הא' אל הב' כיחס הב' אל הג' והנה נכפול הא' על הב' והעולה נכפול על ג' הוא אלף כמה הם המספרים היחסיים |
| word problem/find a number | ⅕a/√a=7 | ספר_דיני_ממונות#VnZO | Question: find me a number, such that when you divide its fifth by a root of the given number, the result is 7.
: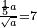 שאלהMS L שיו תמצא לי מספר אשר כשתחלק חמישיתו על שרש המספר המונח שיצא ז'
שאלהMS L שיו תמצא לי מספר אשר כשתחלק חמישיתו על שרש המספר המונח שיצא ז' |
| word problem/find a number | a/b=⁵/₇;(ab)²=a²√8 | ספר_ג'יבלי_אלמוקבאלא#3fAj | Find me two numbers such that the one is a part of the other as 5 is of 7;
:and if the first is multiplied the second, then the product is multiplied by itself, it yields the same as the product of the smaller by itself, then the product multiplied by the root of 8.
:I ask: how much will each number be?
: תמצא לי שני מספרי' שיהיה האחד חלק מהאחר כאשר ה' הוא מז'
תמצא לי שני מספרי' שיהיה האחד חלק מהאחר כאשר ה' הוא מז'ומוכה הראשון בשני ואותה ההכאה תוכה בעצמה יעשה כמו הכאת המספר הקטן בעצמו וההכאה ההיא תוכה בשרש ח' אשאל כמה יבא להיות כל אחד מהמספרי' |
| word problem/find a number | seven proportional numbers | ספר_דיני_ממונות#By9s | 259) Question of seven numbers with fractions: the first is an eighth and the seventh is 91 integers and an eighth. רנט) שאלה משברים מז' מספרים הראשון א' שמינית והז' צ"א שלמים ושמינית |
| word problem/find a number | a²=(3+¹⁰/₁₃)(a+6) | ספר_המספר_/_אליהו_מזרחי#UwCZ | Likewise, if you want to know the number whose square equals the product of three and 10-thirteenths by the sum of that number with 6.
::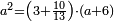 וכן אם רצית לדעת המספר אשר יהיה מרובעו שוה לעולה מהכאת השלשה וי' יגיי"ם עם המספר ההוא מחובר עם ו'
וכן אם רצית לדעת המספר אשר יהיה מרובעו שוה לעולה מהכאת השלשה וי' יגיי"ם עם המספר ההוא מחובר עם ו' |
| word problem/find a number | a/b=¾;a²b=√(20¼) | ספר_ג'יבלי_אלמוקבאלא#4NoH | Find me two numbers such that one is a part of the other as 3 is of 4;
:and when the one is multiplied by itself, and this product is multiplied by the second, it yields a root of 20 and a quarter.
:I ask: how much will each number be?
: עשה לי זה החשבון תמצא לי שני מספרים שיהיה חלק האחד מהאחר כמו מה שהוא ג' מד'
עשה לי זה החשבון תמצא לי שני מספרים שיהיה חלק האחד מהאחר כמו מה שהוא ג' מד'וכשיוכה הראשון בעצמו ואותה ההכאה תכה בשני יעשה שרש כ' ורביע אשאל כמה יבא להיות כל אחד מהמספרים |
| word problem/find a number | b-1=a+1;20(a-1)=b+1 | מלאכת_המספר#osY0 | In order to elaborate the explanation, we give another example: we assume two numbers, such that if we subtract one from the greater number and add it to the smaller, both numbers become equal; and if we subtract one from the smaller number and add it to the greater, the greater becomes twenty times the smaller. [ו]כדי לה[וסי]ף ביאור נעשה משל אחר בשנניח ב' מספר[ים] שאם נחסר אחד מהמספר הגדול [ו]נוסיפהו לקטן יהיה [ה]שני מספרי' שוים ואם נחסר אחד מהמספר הקטן ונוסיפהו בגדול יהיה הגדול עשרי' פעמי' בקטן |
| word problem/find a number | a+½½a+⅓⅓a+⅕⅕a=7 | Arithmetic_Textbook_by_R._Aharon_ben_Isaac#rLug | Question: What is the unknown number, such that when we add to it half of its half, a third of its third, and a quarter of its quarter, it is exactly 7.
: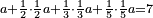 marg.שאלה מהו המספר הנעלם אשר חיברנו עליו חצי חציו ושלישית שלישיתו ורביעית רביעיתו ולא יהיה אלא ז'
marg.שאלה מהו המספר הנעלם אשר חיברנו עליו חצי חציו ושלישית שלישיתו ורביעית רביעיתו ולא יהיה אלא ז' |
| word problem/find a number | a(mod)3=2,a(mod5)=4,a(mod7)=6 | ספר_דיני_ממונות#hx8d | 169) Question: if you are told that the remainder after casting out the threes is 2 etc.
:Do it this way:
:For instance, if it is said that when we divide it by 3, 2 remains; if its is divided by 5, 4 remains; and if it is divided by 7, 6 remains.
קסט) שאלה ואם אמר לך שנשאר בהשליכך ג"ג ב' או ג' עשה בדרך הזה עד"מ ואם אמר כשנחלקהו על ג' ישאר ב' ואם יחולק על ה' ישאר ד' ואם יחולק על ז' ישאר ו' |
| word problem/find a number | a(mod2)=1,a(mod3)=2,a(mod)4=3,a(mod5)=1,a(mod6)=5,a(mod7)=1,a(mod8)=7,a(mod9)=8,a(mod10)=1 | ספר_המספר_/_אליהו_מזרחי#kMUT | Question: if one asks: what is the number that when counted by twos - one remains; by threes - 2 remains; by fours - 3 remains; by fives - one remains; by sixes - five remains; by sevens - one remains; by eights - 7 remains; by nines - eight remains; and by tens - one remains. שאלה אם שאל שואל איזהו המספר אשר כשימנה מב' ב' ישאר אח' ומג' ג' ישארו ב' ומד' ד' ישארו ג' ומה' ה' ישאר אחד ומו' ו' ישארו חמשה ומז' ז' ישאר אחד ומח' ח' ישארו ז' ומט' ט' ישארו שמנה ומי' י' ישאר אחד |
| word problem/find a number | four proportional numbers | ספר_דיני_ממונות#Z5m3 | Example: the first is 2 and the fourth is 16. דמיון המספר הראשון ב' והד' י"ו |
| word problem/find a number | a/b=¾;³√(2a+3b)=8 | ספר_ג'יבלי_אלמוקבאלא#q0Kk | Find me two numbers such that one is a part of the other as 3 is of 4;
:and when the one is multiplied by 2 and the other is multiplied by 3, and the two products are summed together their cube root is 8.
:I ask: how much will each number be?
:![\scriptstyle\begin{cases}\scriptstyle a:b=3:4\\\scriptstyle\sqrt[3]{2a+3b}=8\end{cases}](/mediawiki/images/math/f/d/f/fdfeea3918f71a57781b72d247caef5c.png) והנה המשל עשה לי זה החשבון תמצא לי ב' מספרי'
שיהיה הא' חלק מהאחר כמו שג' הוא מד'
והנה המשל עשה לי זה החשבון תמצא לי ב' מספרי'
שיהיה הא' חלק מהאחר כמו שג' הוא מד'ומוכה הראשון בב' והשני בג' ויקובצו ב' אלו ההכאו' יחד יהיה שרשם המעוקב ח' אשאל כמה יבא להיות כל א' מהמספרי' |
| word problem/find a number | four proportional numbers | ספר_דיני_ממונות#VcKa | Example: the first is 3-quarters and the last is 6 integers. דמיון הראשון ג' רביעיות והאחרון ו' שלמים |
| word problem/find a number | five proportional numbers | ספר_דיני_ממונות#lGXB | 257) Example of five numbers: the first is 3 and the last is 48. רנז) דמיון מה' מספרים הראשון ג' והאחרון מ"ח |
| word problem/find a number | a+b=n², a-b=m² | ספר_דיני_ממונות#U3Ow | 332) Question: find me a number such that when you add a certain number to it, it becomes a square, and if you subtract it from it, a square remains.
: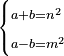 שלב) שאלה תמצא לי מספר אשר כשתוסיף עליו מספר מה יהיה מרובע 284vואם תגרע ממנו ישאר מרובע
שלב) שאלה תמצא לי מספר אשר כשתוסיף עליו מספר מה יהיה מרובע 284vואם תגרע ממנו ישאר מרובע |
| word problem/find a number | a(mod)1=0,a(mod2)=1,a(mod3)=2,a(mod)4=3,a(mod5)=4,a(mod6)=5,a(mod7)=1 | ספר_דיני_ממונות#65rT | 333) Question: find me a number, such that when you count it by ones it is gone; by twos - 1 remains; by threes - 2; by fours - 3; by fives - 4; by sixes - 5; and by sevens - 1. שלג) שאלה תמצא לי מספר כי כשתמנהו א"א שוה ב"ב ישאר א' ג"ג ב' ד"ד ג' ה"ה ד' ו"ו ה' ז"ז א' |
| word problem/find a number | a/b=¾;a²b=288 | ספר_ג'יבלי_אלמוקבאלא#7M0L | Find me two numbers such that the one is a part of the other as 3 is of 4;
:and when the smaller is multiplied by itself, and the product is multiplied by the larger, it yields 288.
:I ask: how much will each number be?
: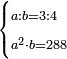 תמצא לי שני מספרים שיהיה האחד חלק מהאחר כמו שהוא ג' מד'
תמצא לי שני מספרים שיהיה האחד חלק מהאחר כמו שהוא ג' מד'וכשיוכה הקטן בעצמו ו{{#annot: term | #result, #יוצא | QU3I}}היוצא{{#annotend:QU3I}} תכה בגדול יעשה רפ"ח אשאל כמה כל אחד מהמספרי' |
| word problem/find a number | (a·⅔a)²=√50 | ספר_ג'יבלי_אלמוקבאלא#MvD8 | Find me a number, such that when it is multiplied by its two thirds, and that product is multiplied by itself, it yields the root of fifty.
:I ask: how much will the number be?
: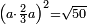 עשה לי זה החשבון תמצא לי מספר אחד שכאשר הוכה בשני שלישיו ואותה ההכאה תוכה בעצמה תעשה שרש חמשים
עשה לי זה החשבון תמצא לי מספר אחד שכאשר הוכה בשני שלישיו ואותה ההכאה תוכה בעצמה תעשה שרש חמשיםאשאל כמה יבא להיות המספר האמור |
| word problem/find a number | perfect number | ספר_דיני_ממונות#CaJp | 110) If you want to find the third [perfect number] קי)MS L: om. ואם ואם תרצה למצא השלישי |
| word problem/find a number | (a+b)\c=3⅖⅐;(a+c)\b=7⅔¼;b=30 | ספר_מעשה_חושב#odgb | Example: when one number is added to a second number, their ratio to the third is 3 integers, 2-fifths and a seventh.
::When the first is added to the third, their ratio to the second is 7 integers, 2-thirds and a quarter.
::The second number is 30.
::We wish to know: how much is each of the rest?
::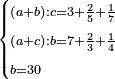 דמיון שיהיה מספר ראשון כשחובר אל מספר שני היה יחסו אל השלישי ג' שלמים וב' חמשיות ושביעית
דמיון שיהיה מספר ראשון כשחובר אל מספר שני היה יחסו אל השלישי ג' שלמים וב' חמשיות ושביעיתוכשחובר הראשון אל השלישי יהיה יחסו אל השניאל השני: P2271 הראשון ז' שלמים וב' שלישיות ורביעית והמספר השני שלשים ורצינו לדעת כמה כל אחד מהנשארים |
| word problem/find a number | 4a/5=6⅗ | ספר_דיני_ממונות#lrPR | Another question: find me a number such that when you multiply it by 4, then divide the product by 5, the result of division is 6 and the remainder is 3.
: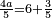 שאלה אחרת תמצא לי מספר אשר כשתכפלהו על ד' ותחלקהו על ה' שיצא ו' בחלוק וישאר ג'
שאלה אחרת תמצא לי מספר אשר כשתכפלהו על ד' ותחלקהו על ה' שיצא ו' בחלוק וישאר ג' |
| word problem/find a number | 9a=1 | Arithmetic_Textbook_by_R._Aharon_ben_Isaac#LF1Q | The seventh question: what is the number, such that when we multiply it by 9, the product is one.
: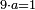 שאלה שביעית מהו המספר שאם כפלנו אותו על ט' והיוציא יהיה אחד
שאלה שביעית מהו המספר שאם כפלנו אותו על ט' והיוציא יהיה אחד |
| word problem/find a number | 8/a/b/27 | ספר_דיני_ממונות#cJJC | Question: if you wish to find two numbers standing between 8 and 27, such that the ratio of the second to the first is as the ratio of the third to the second and as the ratio of the fourth to the third.
: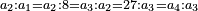 שאלה אם תרצה למצא ב' מספרים יעמדו בין ח' ובין כ"ז שיהיה יחס השני אל הראשון כיחס הג' אל הב' וכיחס הד' אל הג'
שאלה אם תרצה למצא ב' מספרים יעמדו בין ח' ובין כ"ז שיהיה יחס השני אל הראשון כיחס הג' אל הב' וכיחס הד' אל הג' |
| word problem/find a number | a/b=⅖;ab=8√b | ספר_ג'יבלי_אלמוקבאלא#BstD | Find me two numbers such that the one is a part of the other as 2 is of 5;
:and when the first is multiplied by the second, it yields the same as the product of the root of the second by 8.
:I ask: how much will each number be?
: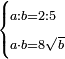 תמצא לי שני מספרי' שיהיה האחד חלק מהאחר כמו שב' הוא מה'
תמצא לי שני מספרי' שיהיה האחד חלק מהאחר כמו שב' הוא מה'וכשהוכה האחד בשני יעשה כמו הכאת שרש השני בח' אשאל כמה יבא להיות כל אחד מהמספרי' |
| word problem/find a number | a/b=⅓;³√a³√b=100 | ספר_ג'יבלי_אלמוקבאלא#AP3O | Find me two numbers such that the one is a part of the second as 1 is of 3;
:and the cube root of the first multiplied by the cube root of the second yields 100.
:I ask: how much will each number be?
:![\scriptstyle\begin{cases}\scriptstyle a:b=1:3\\\scriptstyle\sqrt[3]{a}\sdot\sqrt[3]{b}=100\end{cases}](/mediawiki/images/math/6/b/6/6b684d922156985988350c4b2a4c248d.png) והנה המשל עשה לי זה החשבון תמצא לי 43rשני מספרי' שיהיה הראשון חלק מהשני כמו שא' הוא מג'
והנה המשל עשה לי זה החשבון תמצא לי 43rשני מספרי' שיהיה הראשון חלק מהשני כמו שא' הוא מג'ומוכה שרש מעו' מהראשון בשרש מעו' השני יעשה ק' אשאל כמה יבא להיות כל אחד מן המספרי' |
| word problem/find a number | three proportional numbers | ספר_דיני_ממונות#qdnU | Example: we have three proportional numbers, the first is 2, and the third is 18. דמיון יש לנו ג' מספרים מתיחסים הראשון ב' והג' י"ח |
| word problem/find a number | a(mod)3=a(mod5)=a(mod7)=1 | ספר_דיני_ממונות#lQns | 168) Question: find me a number, such that if you divide it by 3, by 5, and by 7, 1 remains. קסח) שאלה בקש לי מספר שאם תחלקהו על ג' ועל ה ועל ז' ישאר א' |
| word problem/find a number | 4a(mod)5=1 | ספר_דיני_ממונות#3egI | Example from which you can understand all that is mentioned concerning the nativities: it is that you find me a number such that when you multiply it by 4, then divide the product by 5, the remainder is 1.
: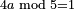 דמיון אשר ממנו תוכל להבין כל הנזכר במולדות
דמיון אשר ממנו תוכל להבין כל הנזכר במולדותוהוא תמצא לי מספר אשר כשתכפלהו על ד' והעולה תחלק על ה' שישאר א' |
| word problem/find a number | a/b=⅔;3a+4b=³√216 | ספר_ג'יבלי_אלמוקבאלא#w4Ac | Find me two numbers such that one is a part of the other as 2 is of 3;
:and when the first is multiplied by 3 and the second by 4, and both products are summed together, they yield a cube root of 216.
:I ask: how much will each number be?
:![\scriptstyle\begin{cases}\scriptstyle a:b=2:3\\\scriptstyle3a+4b=\sqrt[3]{216}\end{cases}](/mediawiki/images/math/3/7/c/37c574e931d2b293ce8e7c05872dd409.png) והנה המשל תמצא לי ב' מספרי' שיהיה האחד חלק מהאחר כמו שב' הוא מג'
והנה המשל תמצא לי ב' מספרי' שיהיה האחד חלק מהאחר כמו שב' הוא מג'ומוכה הראשון בג' והשני בד' ויחוברו ב' אלו ההכאו' יחד יעשה שרש מעוק' מרי"ו אשאל כמה יבא להיות כל א' מהמספרי' |
| word problem/find a number | √(9a)=12 | ספר_ג'יבלי_אלמוקבאלא#TeOu | Find me a number, such that when it is multiplied by 9, the root of the product is 12.
:I ask: how much will the number be?
: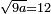 תמצא לי מספר אחד שכאשר יוכה בט' יהיה שרש העולה י"ב
תמצא לי מספר אחד שכאשר יוכה בט' יהיה שרש העולה י"באשאל כמה יבא להיות המספר |
| word problem/find a number | a-⅓a-¼a=29 | ספר_דיני_ממונות#3Zd3 | 135) Question: find me a number such that if you subtract its third and its quarter from it the remainder is 29.
: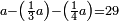 קלה)MS L: קלב שאלה תמצא לי מספר אשר אם תוציא ממנו שלישיתו ורביעיתו הנשאר כ"ט
קלה)MS L: קלב שאלה תמצא לי מספר אשר אם תוציא ממנו שלישיתו ורביעיתו הנשאר כ"ט |
| word problem/find a number | a²-5a=n², a²+7a=m² | ספר_דיני_ממונות#3YCC | 158) Question: if you want to find a square number such that if you subtract its five roots from it the remainder is a square and if you add it to its seven roots it will be a square.
: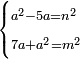 קנח)MS L: קנה שאלה אם תרצה לדעת מספר אחד מרובע אשר אם תוציא אותו מה' שרשיו וישאר מרובע ואם תוסיף עליו שבעה שורשיו יהיה מרובע
קנח)MS L: קנה שאלה אם תרצה לדעת מספר אחד מרובע אשר אם תוציא אותו מה' שרשיו וישאר מרובע ואם תוסיף עליו שבעה שורשיו יהיה מרובע |
| word problem/find a number | ³/₇a+⁴/₅a=⅔a+¼a+20 | ספר_מעשה_חושב#7YKC | Example: three-sevenths and four-fifths of the unknown number exceed two-thirds and a quarter of the unknown number by twenty and we wish to know how much is the number?
::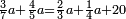 דמיון זה שיהיו ג' שביעיות וד' חמישיות המספר הנעלם מוסיפים על ב' שלישיות ורביעית המספר הנעלם עשרים ורצינו לדעת כמה המספר
דמיון זה שיהיו ג' שביעיות וד' חמישיות המספר הנעלם מוסיפים על ב' שלישיות ורביעית המספר הנעלם עשרים ורצינו לדעת כמה המספר |
| word problem/find a number | a/b=⅔;4a+4b=√100 | ספר_ג'יבלי_אלמוקבאלא#FMUp | Find me two numbers such that one is a part of the other as 2 is of 3;
:and when each of them is multiplied by 4, and both products are summed together, they yield a root of 100.
:I ask: how much will each number be?
: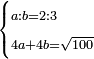 תמצא לי שני מספרים שיהיה חלק האחד מהאחר כמו שהוא ב' מג'
תמצא לי שני מספרים שיהיה חלק האחד מהאחר כמו שהוא ב' מג'וכשיוכה כל אחד בארבעה ויקובצו אלו ההכאות יחד יעשו שרש ק' אשאל כמה יבא להיות כל מספר מהם |
| word problem/find a number | a/b=⅔;a²b=b√b | ספר_ג'יבלי_אלמוקבאלא#DcQa | Find me two numbers such that the one is a part of the other as 2 is of 3;
:and when the first is multiplied by itself and this product is multiplied by the second, it yields the same as the product of the second by its root.
:I ask: how much will each number be?
: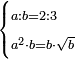 תמצא לי שני מספרי' שיהיה האחד חלק מהשני כמו שב' הוא מג'
תמצא לי שני מספרי' שיהיה האחד חלק מהשני כמו שב' הוא מג'ומוכה הראשון בעצמו ואותה ההכאה תוכה במספר השני יעשה 34vכמו הכאת השני בשרשו אשאל כמה יבא להיות כל אחד מהמספרי' |
| word problem/find a number | a/b=⅗;ab=³√729 | ספר_ג'יבלי_אלמוקבאלא#ai8Z | Find me two numbers such that one is a part of the other as 3 is of 5;
:and the one multiplied by the other yields a cube root of 729.
:I ask: how much will each number be?
:![\scriptstyle\begin{cases}\scriptstyle a:b=3:5\\\scriptstyle a\sdot b=\sqrt[3]{729}\end{cases}](/mediawiki/images/math/0/5/a/05a756d12f1bd790b4653042daf26458.png) והנה המשל תמצא לי ב' מספרי' שיהיה האחד חלק מהאחר כמו ג' מה'
והנה המשל תמצא לי ב' מספרי' שיהיה האחד חלק מהאחר כמו ג' מה'ומוכה הראשון בשני יעשה שרש מעו' מתשכ"ט אשאל כמה יבא להיות כל אחד מהמספרי' |
| word problem/find a number | (a+b)/c=⅗+⅙;(a+c)/b=2⅓;a=20 | ספר_דיני_ממונות#gfLj | 59) Question: the ratio of the first and the second summed together to the third is 3-fifths plus a sixth.
:The ratio of the first and the third summed together to the second is 2 integers and a third.
:The first number is 20.
:We wish to know: how much is each of the remaining numbers?
נט) שאלה יהיה יחס הראשון עם השני מקובצים אל השלישי ג' חמישיות ושישית ויחס הראשון והג' מקובצים אל השני ב' שלמים ושלישית והמספר הראשון עשרים נרצה לדעת כמה כל א' מהמספרים הנשארים |
| word problem/find a number | a/b=¾;8a=6√b | ספר_ג'יבלי_אלמוקבאלא#8HYy | Find me two numbers such that the one is a part of the second as 3 is of 4;
:and the first multiplied by 8, yields the same as the root of the second multiplied by 6.
:I ask: how much will each number be?
: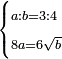 תמצא לי שני מספרי' שיהיה החלק האחד מהשני כמו שהוא ג' מד'
תמצא לי שני מספרי' שיהיה החלק האחד מהשני כמו שהוא ג' מד'וכן יעשה הראשון מוכה בח' כמו השני דהיינו שרשו מוכה בו' אשאל כמה יבא להיות כל אחד מהמספרים |
| word problem/find a number | ³/₇a+⁴/₅a=⅔a+¼a+20 | ספר_דיני_ממונות#SkQW | 46) Question: three sevenths and four fifths of the unknown number exceed two thirds and a quarter of the unknown number by twenty.
:We wish to know: how much is the number?
: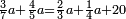 מו) שאלה ג' שביעיות וד' חמישיות המספר הנעלם מוסיפים על ב' שלישיות ורביעית המספר הנעלם כ'
מו) שאלה ג' שביעיות וד' חמישיות המספר הנעלם מוסיפים על ב' שלישיות ורביעית המספר הנעלם כ'רצינו לדעת כמה המספר הנעלם |
| word problem/find a number | ⅓a+⅕a=10 | מלאכת_המספר#niUj | Suppose we want to find any whole, such that the sum of its third and its fifth together is ten.
: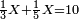 נניח שרצינו למצא אי זה כל שקבוץ שלישיתו וחמישיתו יחד הם עשרה
נניח שרצינו למצא אי זה כל שקבוץ שלישיתו וחמישיתו יחד הם עשרה |
| word problem/find a number | a(mod2)=1,a(mod3)=2,a(mod)4=3,a(mod5)=4,a(mod6)=5,a(mod7)=0 | ספר_המספר_/_אליהו_מזרחי#jLBc | Question: if one asks: what is the number that when counted by twos - one remains; by threes - 2 remains; by fours - 3 remains; by fives - 4 remains; by sixes - 5 remains; and by sevens - nothing remains. שאלה אם שאל שואל איזהו המספר אשר כשימנה מב' ב' ישארו א' ומג' ג' ישארו ב' ומד' ד' ישארו ג' ומה' ה' ישארו ד' ומו' ו' ישארו ה' ומז' ז' לא ישארו כלום |
| word problem/find a number | a+¼(b+c)=b+⅙(a+c)=c+⅑(a+b);b=20 | ספר_דיני_ממונות#HxDL | 62) Question: the first with a quarter of the rest is equal to the second with a sixth of the rest and is also equal to the third with a ninth of the rest.
:It has already been explained to you in the first section of this book that the order of these three numbers is according to this arrangement, meaning that the first is added to the greatest part of the rest and the [third] is added to the smallest part of the rest. I remind you this so that you will not be confused by the explanations.
:The second number is 20.
:We wish to know: how much is each of the rest?
סב) שאלה שיהיה הראשון עם רביעית הנשארים שוה אל השני עם שישית הנשארי' והוא ג"כ שוה אל השלישי עם תשיעית הנשארים כבר התבאר לך במאמ' הראשון מזה הספר שסדור אלו המספרים הג' הוא על זה הסדר אשר סדרוה רצוני שהראשון הוא אשר יתחבר המתחלק יותר גדול מן הנשארים והל' הוא אשר יתחבר עמו יותר קטן והנשארים והזכרתי זה לך למען לא תבלבל בביאורים והנה יהיה המספר השני מהם כ' ורצינו לדעת כמה כל א' מהנשארים |
| word problem/find a number | (½a+⅓a)-(¼a+⅕a)=4 | ספר_דיני_ממונות#cQy5 | Question: find me a number, such that [the sum of] its half and its third exceeds [the sum of] its quarter and its fifth by 4 integers.
: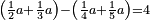 שאלהMS L שו תמצא לי מספר אשר מחציתו ושלישיתו יוסיפו ד' שלמים על רביעיתו וחמישיתו
שאלהMS L שו תמצא לי מספר אשר מחציתו ושלישיתו יוסיפו ד' שלמים על רביעיתו וחמישיתו |
| word problem/find a number | ⅓a+¼a=6 | מלאכת_המספר#m65t | Example: we wish to find a number, whose quarter and third are 6.
: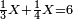 המשל רצינו למצא מספר אחד שרביעיתו ושלישיתו יהיה ו'
המשל רצינו למצא מספר אחד שרביעיתו ושלישיתו יהיה ו' |
| word problem/find a number | 4=⅓·15→a=⅕·25 | ספר_דיני_ממונות#RldY | 65) Question: if number 4 is a third of number 15 what is the fifth of 25? סה) שאלה אם מספר ד' הוא שליש מספר ט"ו מהו חומש כ"ה |
| word problem/find a number | ⅖a²=a | ספר_דיני_ממונות#LWXU | 159) Quaestion: if you want to find a number such that its two fifths are its root.
: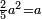 קנט)MS L: קנו שאלה אם תרצה למצא מספר אשר ב' חמישיות שלו יהיה שרשו
קנט)MS L: קנו שאלה אם תרצה למצא מספר אשר ב' חמישיות שלו יהיה שרשו |
| word problem/find a number | a-⅓a=√a | ספר_האלזיברא#HmbP | Question: I want to find a number such that when its third is subtracted from it the remainder is the root of the original number
: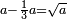 שאלה רציתי למצא מספר כאשר {{#annot: term | #to subtract, #חסר | rg2F}}חסר ממנו{{#annotend:rg2F}} שלישית הנשאר הוא שרש המספר כלו
שאלה רציתי למצא מספר כאשר {{#annot: term | #to subtract, #חסר | rg2F}}חסר ממנו{{#annotend:rg2F}} שלישית הנשאר הוא שרש המספר כלו |
| word problem/find a number | a/b=⅔;(3a+4b)√5=40 | ספר_ג'יבלי_אלמוקבאלא#PB7l | Find me two numbers such that one is a part of the other as 2 is of 3;
:and when the first is multiplied by 3 and the second by 4, and both products are summed together and multiplied by the root of 5, it yields 40.
:I ask: how much will each number be?
: והנה המשל תמצא לי שני מספרים שיהיה חלק אחד מהם לאחר כמו שהוא ב' אל ג'
והנה המשל תמצא לי שני מספרים שיהיה חלק אחד מהם לאחר כמו שהוא ב' אל ג'וכשיוכה הראשון בשלשה והשני בארבעה ואלו שתי ההכאות מחוברים יחד ויוכה בשרש ה' יעשה מ' אשאל כמה יבא להיות כל אחד מהמספרי' |
| word problem/find a number | a/b=⅗;a²b=b²√8 | ספר_ג'יבלי_אלמוקבאלא#YrhT | Find me two numbers such that the one is a part of the other as 3 is of 5;
:and the the first is multiplied by the other, then this product is multiplied by the second number, it yields the same as the product of the second by itself, then the product is multiplied by the root of 8.
:I ask: how much will each number be?
: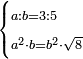 עשה לי זה החשבון תמצא לי שני מספרי' שיהיה האחד חלק מהאחר כמו שג' הם מה'
עשה לי זה החשבון תמצא לי שני מספרי' שיהיה האחד חלק מהאחר כמו שג' הם מה'ומוכה הראשון בעצמו ואותה ההכאה תוכה אשאל כמה יבא להיות כל אחד מהמספרי' |
| word problem/find a number | a+⅓a+⅖(a+⅓a)+¾(a+⅓a+⅖(a+⅓a))=13 | Arithmetic_Textbook_by_R._Aharon_ben_Isaac#KQWm | Question two: What is the number, such that if you add to it its third, then to the sum 2-fifths of the sum, then to this sum 3-quarters of the sum, [and after all this the result is 13]. שאלה שנית מהו המספר אשר אם תחבר עליו שלישיתו ועל העולה ב' חמשיות מן העולה ועל העולה ג' רביעיות מן [97v]העולה [ ועם כל זה יעלה י"ג] |
| word problem/find a number | (3a/4)/4=4/6 | ספר_דיני_ממונות#mnbD | 43) Question: find me a number such that when multiplied by 3 then divided by 4 the ratio of the remainder to 4 is the same as the ratio of 4 to 6.
: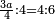 מג) שאלה תמצא לי מספר אשר כשתכפול על ג' ותחלק על ד' ומה שישאר יהיה יחסו אל ד' כיחס ד אל ו'
מג) שאלה תמצא לי מספר אשר כשתכפול על ג' ותחלק על ד' ומה שישאר יהיה יחסו אל ד' כיחס ד אל ו' |
| word problem/find a number | a/b=⅔;ab=√12 | ספר_ג'יבלי_אלמוקבאלא#zNTn | Find me two numbers such that one is a part of the other as 2 is of 3;
:and if one is multiplied by the other, it yields a root of 12.
:I ask: how much will each number be?
: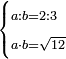 תמצא לי שני מספרים שיהיה חלק האחד מהאחר כמו שהוא ב' מג'
תמצא לי שני מספרים שיהיה חלק האחד מהאחר כמו שהוא ב' מג'ואם יוכה האחד באחר יעשה שרש מי"ב אשאל כמה יבא להיות כל אחד מהמספרים |
| word problem/find a number | a+4=n², a-4=m² | ספר_דיני_ממונות#bQqS | 151) Question: if you want to find a number such that if you add to it 4 it will be a square and if you subtract [from it 4] it will also be a square.
: קנא)MS L: קמח שאלה אם תרצה למצא מספר אשר אם תוסיף עליו ד' יהיה מרובע ואם תחסר ג"כ יהיה מרובע
קנא)MS L: קמח שאלה אם תרצה למצא מספר אשר אם תוסיף עליו ד' יהיה מרובע ואם תחסר ג"כ יהיה מרובע |
| word problem/find a number | a/b=⅔;5a+7b=16+√8 | ספר_ג'יבלי_אלמוקבאלא#qQCL | Find me two numbers such that the one is a part of the other as 2 is of 3;
:and when the first is multiplied by 5 and the second by 7, then both the products are summed together, it yields 16 and a root of 8.
:I ask: how much will each number be?
: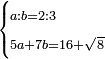 תמצא לי שני מספרי' שיהיה האחד מהאחר כמו שב' הוא מג'
תמצא לי שני מספרי' שיהיה האחד מהאחר כמו שב' הוא מג'ומוכה הראשון בה' והשני בז' ושתי אלו ההכאות יחוברו יחד יעשו י"ו ושרש ח' אשאל כמו' יבא להיות כל אחד מהמספרי' |
| word problem/find a number | a/b=⅔;(ab)²=b√8 | ספר_ג'יבלי_אלמוקבאלא#6dSA | Find me two numbers such that the first is a part of the second as 2 is of 3;
:and when the smaller is multiplied by the greater, and this product is multiplied by itself, it yields the same as the product of the second by the root of 8.
:I ask: how much will each number be?
: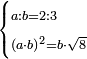 תמצא לי שני מספרים שיהיה הראשון חלק מהשני כמו שהוא ב' מג'
תמצא לי שני מספרים שיהיה הראשון חלק מהשני כמו שהוא ב' מג'ויוכה הקטון בגדול ואותה ההכאה תוכה בעצמה יעשה כמו הכאת השני בשרש ח' אשאל כמה יבא להיות כל אחד מהמספרי' |
| word problem/find a number | a/b=⅔;(ab)b=100+\sqrt{8} | ספר_ג'יבלי_אלמוקבאלא#GBTu | Find me two numbers such that the first is a part of the other as 2 is of 3;
:and when the first is multiplied by the second, then this product is multiplied by the second, it yields 100 and a root of 8.
:I ask: how much will each number be?
: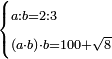 עשה לי החשבון תמצא לי שני מספרים שיהיה המספר ראשון חלק מהאחר כמו שב' הוא מג'
עשה לי החשבון תמצא לי שני מספרים שיהיה המספר ראשון חלק מהאחר כמו שב' הוא מג'ובהכות הראשון בשני ואותה ההכאה תוכה בשני יעשה מאה ושרש ח' אשאל כמה יבא להיות כל אחד מהמספרי' |
| word problem/find a number | 2(b-1)=a+1;3(a-1)=b+1 | מלאכת_המספר#9M9M | The relation of two numbers such that if the greater gives one to the smaller, the smaller becomes double the greater; and if the smaller gives one to the greater, the greater becomes three times the smaller ==== |style="width:45%; text-align:right;"|הפרק הג' ביחס שני מספרי' שאם הגדול יתן אחד לקטן יהיה הקטן כפל הגדול ואם הקטן יתן אחד לגדול יהיה הגדול שלשה פעמי' יותר מהקטן |
| word problem/find a number | 7a/9=45 | ספר_דיני_ממונות#d4nh | 133) Question: find me a number such that if you multiply it by 7 and divide by 9, your result will be 45.
: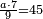 קלג)MS L: קל שאלה תמצא לי מספר אשר אם תכה אותו בז' ותחלק אותו על ט' שיצא לך מ"ה
קלג)MS L: קל שאלה תמצא לי מספר אשר אם תכה אותו בז' ותחלק אותו על ט' שיצא לך מ"ה |
| word problem/find a number | (5/a)·8=23 | ספר_דיני_ממונות#km4W | 134) Question: find a number such that if you divide it by 5 and multiply by 8 the result will be 23.
: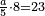 קלד)MS L: קלא שאלה תמצא מספר אשר אם תחלק אותו עם ה' ותכה אותו עם ח' יעלה כ"ג
קלד)MS L: קלא שאלה תמצא מספר אשר אם תחלק אותו עם ה' ותכה אותו עם ח' יעלה כ"ג |
| word problem/find a number | b/(c-a)=3⅓;c/(b-a)=6 | ספר_דיני_ממונות#73tk | 60) Question: the ratio of the second to what remains from the third when the first is subtracted from it is 3 integers and a third.
:The ratio of the third to what remains from the second when the first is subtracted from it is the number 6.
[ס)] שאלה שיהיה יחס הב' אל הנשאר מהג' כשחוסר ממנו הא' ג' שלמים ושליש ויחס הג' אל הנשאר מהב' כשחוסר ממנו הא' מספר ו' |
| word problem/find a number | (a·⅔a)²=36 | ספר_ג'יבלי_אלמוקבאלא#IqWv | Find me a number, such that when it is multiplied by its two thirds, and the product is multiplied by itself, it yields 36.
:I ask: how much will the number be?
:![\scriptstyle\left[a\sdot\left(\frac{2}{3}\sdot a\right)\right]^2=36](/mediawiki/images/math/4/4/d/44d26474e128ec5582fe301dd2b9863a.png) תמצא לי מספר אחד שכשיוכה בשני שלישיו ועולה שני שלישי צינסו והעולה יוכה בעצמו
יעשה ל"ו
תמצא לי מספר אחד שכשיוכה בשני שלישיו ועולה שני שלישי צינסו והעולה יוכה בעצמו
יעשה ל"ואשאל כמה יבא להיות המספר |
| word problem/find a number | a²·¾a²=6a | ספר_ג'יבלי_אלמוקבאלא#GJDy | Find me a number, such that when it is multiplied by itself, and the product is multiplied by its three quarters, it yields the same as 6 times the number.
:I ask: how much will this number be?
: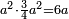 תמצא לי מספר אחד שכאשר הוכה בעצמו ומה שיעלה יוכה ב'''שני שלישיו''' יעשה כמו ו' דמיוני המספר האמור
תמצא לי מספר אחד שכאשר הוכה בעצמו ומה שיעלה יוכה ב'''שני שלישיו''' יעשה כמו ו' דמיוני המספר האמוראשאל כמה יהיה המספר האמור |
| word problem/find a number | ½a+⅓a+¼a-5=24 | Arithmetic_Textbook_by_R._Aharon_ben_Isaac#ywwH | Second question: What is the number whose half, third and quarter, minus five are 24?
: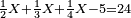 שאלה שנית מהו המומן הנעלם אשר חציו ושלישיתו ורביעיתו פחות חמשה יהיו כ"ד
שאלה שנית מהו המומן הנעלם אשר חציו ושלישיתו ורביעיתו פחות חמשה יהיו כ"ד |
| word problem/find a number | (a+b)/c=⅗+⅙;(a+c)/b=2⅓;a=20 | ספר_מעשה_חושב#0XvG | Another example: the ratio of the first and the second summed together to the third is 3-fifths and a sixth.
::The ratio of the first and the third summed together to the second is 2 integers and a third.
::The first number is 20.
::We wish to know: how much is each of the remaining numbers?
::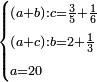 דמיון אחר יהיה יחס הראשון והשני מקובצים אל השלישי ג' חמשיות וששית
דמיון אחר יהיה יחס הראשון והשני מקובצים אל השלישי ג' חמשיות וששיתויחס הראשון והשלישי מקובצים אל השני ב' שלמים ושלישית והמספר הראשון עשרים ורצינו לדעת כמה כל אחד מ[ה]מספרים הנשארים |
| word problem/find a number | a²-15=b² | ספר_דיני_ממונות#eiGo | 150) Question: if you want to find a square number such that if you subtract 15 from it, it remains a square.
: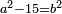 קנ)MS L: קמז שאלה אם תרצה למצא מרובע שאם תוציא ממנו ט"ו ישאר מרובע
קנ)MS L: קמז שאלה אם תרצה למצא מרובע שאם תוציא ממנו ט"ו ישאר מרובע |
| word problem/find a number | a²=b-5;a²+b²=100 | ספר_ג'יבלי_אלמוקבאלא#a55J | Find me two numbers, such that when the first is multiplied by itself it yields the second minus 5, and when each of them is multiplied by itself, then both products are summed together, it yields 100.
:I ask: how much will each of the numbers be?
: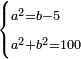 ואשים לך המשל ואומ' כן עשה לי זה החשבון תמצא לי שני מספרי' שכאשר יוכה הראשון בעצמו יעשה השני פחות ה'
ואשים לך המשל ואומ' כן עשה לי זה החשבון תמצא לי שני מספרי' שכאשר יוכה הראשון בעצמו יעשה השני פחות ה'ויוכו כל אחד מהם בעצמו ויחוברו אותם ההכאות יחד יעשה ק' ואשאל יבא כמה להיות כל אחד מהמספרים |
| word problem/find a number | a/b=⅗;ab√8=b | ספר_ג'יבלי_אלמוקבאלא#nOEQ | Find me two numbers such that the one is a part of the other as 3 is of 5;
:and when the one is multiplied by the other, then this product is multiplied by the the root of 8, it yields the same as the second number.
:I ask: how much will each number be?
: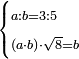 עשה לי זה החשבון תמצא לי ב' מספרי' שיהיה האחד חלק מהאחר כמו שהוא ג' מה'
עשה לי זה החשבון תמצא לי ב' מספרי' שיהיה האחד חלק מהאחר כמו שהוא ג' מה'וכשיוכה האחד באחר ואותה ההכאה תוכה בשרש ח' יעשה כמו המספר השני אשאל כמה הוא כל אחד מהמספרים |
| word problem/find a number | five proportional numbers | ספר_דיני_ממונות#BxZ9 | [2]52) Question: if you have 5 proportional numbers, the first is 3 and the fifth is 768, what is the second?
קנב) שאלה אם יש לך ה' מספרים מתיחסים הא' ג' והה' תשס"ח מהו השני |
| word problem/find a number | ⅓a+¼a=10½ | מלאכת_המספר#IQCS | Example for this: suppose the sum of a third and a quarter of the whole is equal to ten and a half and we wish to know the whole.
: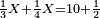 המשל לזה נניח קבוץ אחד משליש ורביע ששוה עשרה וחצי ורצינו לדעת הכל
המשל לזה נניח קבוץ אחד משליש ורביע ששוה עשרה וחצי ורצינו לדעת הכל |
| word problem/find a number | ¼a+⅕a=5 | מלאכת_המספר#0Nd6 | As the one who asks about the sum of a quarter and a fifth [of a whole], which is 5 integers, and we wish to know how much is the whole.
: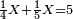 כמי שישאל על קבוץ רביע וחומש שהם ה' שלימי' ונרצה לדעת הכל כמה הוא
כמי שישאל על קבוץ רביע וחומש שהם ה' שלימי' ונרצה לדעת הכל כמה הוא |
| word problem/find a number | a(mod2)=1,a(mod3)=2,a(mod)4=3,a(mod5)=0 | ספר_המספר_/_אליהו_מזרחי#iIDQ | Likewise: if one asks: what is the number that when counted by twos - one remains; by threes - two remains; by fours - three remains; and by fives - nothing remains. וכן אם שאל שואל איזהו המספר אשר כשימנה מב' ב' ישארו אחד ומג' ג' ישארו שנים ומד' ד' ישארו שלשה ומה' ה' לא ישאר דבר |
| word problem/find a number | a(mod2)=0,a(mod3)=2,a(mod)4=0,a(mod5)=0,a(mod6)=2,a(mod7)=3,a(mod8)=4,a(mod9)=5,a(mod10)=0 | ספר_המספר_/_אליהו_מזרחי#lEfW | Likewise: if one asks: what is the number that when counted by twos - nothing remains; by threes - 2 remains; by fours - nothing remains; by fives - nothing remains; by sixes - two remains; by sevens - three remains; by eights - four remains; by nines - five remains; and by tens - nothing remains. וכן אם שאל שואל איזהו המספר אשר כשימנה מב' ב' לא ישאר דבר ומג' ג' ישארו שנים ומד' ד' לא ישאר דבר וחמשה חמשה לא ישאר דבר ומששה ששה ישארו שנים ומז' ז' ישארו שלשה ומח' ח' ישארו ארבעה ומט' ט' ישארו חמשה ומי' לא ישאר דבר |