ספר מעשה חושב
Contents
[hide]- 1 Prologue
- 2 Introduction to Section One – basic definitions
- 3 Section One
- 4 Section Two
- 4.1 Introduction to the Section
- 4.2 Chapter One on the Addition of Numbers to one another and the Subtraction of Numbers from one another
- 4.3 Chapter Two on the Addition of Equal Numbers, which is the Multiplication of a Number by a Number
- 4.4 Chapter Three – Summing Successive or Proportional Numbers
- 4.5 Chapter Four – On the Number of Collections of Given Items that Either Differ by the Items or by their Order or by both
- 4.6 Chapter Five – Division
- 4.7 Chapter Six – Ratios
- 5 Word Problems
- 5.1 Find a Number Problems
- 5.2 Pricing Problems - Find the Price
- 5.3 Conversion: day-hours, liṭra-dinar-pašuṭ
- 5.4 Motion Problems
- 5.5 Motion Problems - Pursuit
- 5.6 Shared Work Problems
- 5.7 Pricing Problems
- 5.8 Purchase Problems - Equal Amounts
- 5.9 Purchase Problems - Unequal Amounts
- 5.10 Payment Problem
- 5.11 Buy and Sell Problems
- 5.12 Barter Problem
- 5.13 Give and Take Problem
- 5.14 Barter Problem
- 5.15 Give and Take Problems
- 5.16 Find a Number Problems
- 6 Colophon
- 7 Notes
- 8 Appendix I: Glossary of Terms
- 9 Appendix II: Bibliography
| ספר מעשה חושב | |
| להחכם הפילוסוף האלקי ר' | |
| לוי בר גרשום | |
Prologue |
|
| Levi ben Gershom said: | [1]אמר לוי בן גרשום |
| Since the complete perfection in a practical craft consists on knowing, in addition to the way of executing each craft, why it is done in this way, and since the practical part of arithmetic is one of the practical crafts, it is clear that we should investigate its reasons. | בעבור שההשגה השלמה בעשיית המלאכה היא שנדע במלאכה מלאכה עם ידיעת אופן המעשה בה למה נעשה אותה בזה האופן והיה החלק המעשי ממלאכת המספר אחת מהמלאכות המעשיות [הוא מבואר][2] שראוי שנחקר בה בסבותיה |
| Another reason compels us to investigate the reasons of this science: It is clear that this science encompasses many types of operations and that each type deals with most varied materials, so that one could think that they do not belong to one type of operation. | ועוד סבה אחרת תחייב [לחקור בזאת המל]אכה[3] בנתינת הסבות וזה שהוא מבואר שזאת המלאכה מ[קפת במינים רבים מאד][4] וכל מין ומין ממנה מקיף בחמרים רבים מתחלפים [התחלפות רב יביא][5] לחשוב שאינם תחת מין אחד |
| Hence, it is clear that the perfection in this science can be complete without knowing the the reasons only with the greatest difficulty. | ובהיות הענין כן הוא מבואר שלא תשלם ההשגה בזאת המלאכה בזולת ידיעת הסבות כי אם בקושי גדול |
| But with the knowledge of the reasons it can be complete easily, for whoever knows the reasons knows with one knowledge the property of the operation in many ways, the execution of which has one and the same reason, while whoever does not know the reasons needs to have a most varied of knowledge in one and the same knowledge, depending on the diversity of the materials. | ואולם עם ידיעת הסבות אפשר שתשלם בקלות והיה זה כן לפי שמי שידע הסבות ידע בידיעה אחת תכונת המעשה במינים רבים אשר תקיף במלאכותיהם סבה אחת בעינה ומי שיסכל הסבות יצטרך בידיעת מלאכה אחת בעינה לידיעות רבות לפי השתנות החמרים |
| Accordingly, we have considered it right to give a brief exposition of the properties of numbers and their reasons in this work. | וכאשר היה זה כן ראינו בזה הספר להודיע דרכי המספרים בסבותיהם לפי קצורנו |
| We have divided this book, according to this investigation, into two sections: | וחלקנו זה הספר לפי זאת החקירה לשני מאמרים |
| The first section contains the fundamentals that apply to what we want to explain about this science. | המאמר הראשון יקיף על השרשים אשר נתן למה שנרצה לבארו מזאת המלאכה |
| The second section deals with the methods of the science for each type of arithmetic operation and giving the reasons. | המאמר השני יקיף על דרכי המלאכה במין מין ממיני המספר ונתינת הסבות |
| Since this work discusses comprehensively both the practice [= maʽase] and the speculation [= ḥoshev], we called it Maʽase Ḥoshev [meaning: the practice of an arithmetician, as a pun on a biblical phrase – "the work of a skilful workman" (Exodus 26, 1; 31; and more)] | ולפי שהיה זה הספר[6] מקיף על המעשה והעיון קראנוהו מעשה חושב |
| Yet, before learning the teaching of the book, he who studies it should precede the study of the 7th, 8th and 9th books of Euclid, for it is not our intention to repeat his words in this book, we will rather set them at the level of principles, since they are already explained there with proofs. | ואולם מדרגת הלמוד הנעשה בזה הספר הנה ראוי שיקדם העיון למעין[7] בו במאמר השביעי והשמיני והתשיעי מאקלידס כי לא היה רצוננו להשיב בזה הספר דבריו[8] אבל נניחם במדרגת השרשים אחר שכבר התבארו שם במופת |
Introduction to Section One – basic definitions |
פתיחת המאמר הראשון |
|
המספר המורכב ממספרים מה הוא כשהוכה הראשון בשני והעולה על השלישי וכן עד כלותם |
|
מספר המספרים והחלקים המונחים הוא מספר מה שבהם ממספרים או מהחלקים [9]מונחים |
|
ויחס המחובר ממספרים מה מונחים אל מספרים [מה מונחים][10] הוא יחס המחובר מיחס הראשון מהקודמים אל הראשון מהנמשכים [ומיחס][11] השני מהקודמים אל השני מהנמשכים וכן עד כלותם |
|
המספרים הנמשכים מתחילים מן האחד הם אחד ושנים ושלשה וכן מה שהגיע ההמשך |
|
המספר הנמשך למספר מה לפניו הוא מה שיחסר מהמספר ההוא אחד |
|
המספר הנמשך למספר מה לאחריו הוא מה שיוסיף על המספר ההוא אחד |
|
נקבץ הנמשכים בדרך המספר מתחילים מן האחד הוא כשחובר אחד עם שנים ועם שלשה וכן מה שהגיע |
|
נקבץ הנפרדים הנמשכים בדרך המספר מתחילין מן האחד הוא כשחובר אחד עם שלשה ועם חמשה וכן מה שהגיע |
|
נקבץ הזוגות הנמשכים בדרך המספר הוא כשחובר שנים שהוא הזוג הראשון עם ארבעה ועם ששה וכן מה שהגיע |
|
המספרים הנמשכים בזולת דרך המספר הוא שיהיה השני מוסיף על הראשון בשיעור מה שיוסיף השליש[י] על השני וכן מה שהגיע |
|
| |
|
והמשך חבור נקבצי הנמשכים בדרך המספר נמשכים בראשיהם ומתחילים מן האחד הם נקבצי הנמשכים[12] אשר תכליתם אחת והראשון מהנקבצים מתחיל מן האחד והשני משנים וכן לא יסורו נמשכים בראשיתם עד התכלית |
|
| |
|
חבור נקבצי הנמשכים בדרך המספר נמשכים בתכליתם ומתחילים מן האחד הם נקבצי הנמשכים אשר כל אחד מהם מתחיל מן האחד[13] והאחד מהם הוא אחד לבד והשני נקבץ אחד ושנים והשלישי נקבץ אחד ושנים ושלשה וכן לא יסורו נמשכים באחרית עד התכלית וכן מה שהגיע |
|
המספר יהיה אמצעי בין מספר מונח ובין האחד אם היה המספר המונח מוסיף עליו בשעור מה שהוא מוסיף על האחד |
|
והמספר המונח קרא הקצווי לזה המספר האמצעי |
|
מיני המספר הם הזוג והנפרד |
|
החלק היותר גדול המספר אשר הוא נקרא בו הוא יותר קטן |
|
והמשל שחצי הוא יותר גדול מחומש והמספר אשר נקרא בו חצי הוא שנים והוא קטן מחמשה אשר נקרא בו חומש |
|
וכבר אפשר שנראה זה במופת בשנניח מספר מה והוא א' ויהיו החלקים ממנו מספר ב' ומספר ג' ויהיה מספר ב' יותר גדול ממספר ג' |
|
ויהיה מספר ד' המספר [14][הקורא לחלק][15] הנקרא בב' ממספר א' |
|
ויהיה מספר ה' המספר הקורא לחלק הנקרא [בג' מ]מספר[16] א' |
|
ואומר שמספר ד' יותר קטן ממספר ה' |
|
המופת כי מפני שמספר ד' הוא המספר הקורא לחלק הנקרא בב' ממספר א' הנה ד' יוכה בב' ויהיה א' |
|
וכזה יתבאר שמספר ג' יוכה בה' ויהיה א' |
|
ואם כן ב' בד' כמו ג' בה' |
|
הנה אם כן צלעותיהם מספיקות יחס ב' אל ג' כיחס ה' אל ד' |
|
אבל |
|
והוא מה שרצינו לבאר |
|
החלק או נקבץ החלקים יהיה גדול מחלק או מנקבץ החלקים אם יהיה החלק ההוא או נקבץ החלקים ההם ממספר מה יותר גדול מהחלק האחר או נקבץ החלקים האחר הלקוח מהמספר ההוא בעינו |
|
יקרה לאחד החלוקה מצד הנושא והוא צד אחר מהעיון באחד מספר המופשט מנושא אבל זה הספר מקיף בשני הענינים יחד ולזה לא נחוש אם יחלק האחד בקצת תמונות המאמר הראשון |
Section One |
המאמר הראשון |
| It encompasses the foundations given for this craft. | והוא מקיף על השרשים אשר נתן בזאת המלאכה |
Theorems in Euclidean style |
|
|
א השטח ההוה מהכאת שני מספרים האחד באחד ימנהו כל מספר מהם במנין אחדי המספר השני[18] |
|
ב כאשר היו שני מספרים מונחים וחולק המספר האחד לחלקים כמה שיהיו הנה שטח המספר האחד בשני שוה לשטחי כל אחד מחלקי המספר האחד בשני מקובצים |
|
ויהיו המספרים המונחים מספר א"ב ג' |
|
וחולק מספר א"ב לחלקים א"ה ה"ד ד"ב |
|
ואומר ש שטח א"ב בג' שוה לשטח א"ה בג' ולשטח ה"ד בג' ולשטח ד"ב[19] בג' מקובצים |
|
המופת ששטח א"ה בג' ימנהו ג' במנין מה שבא"ה מן האחדים |
|
ושטח ה"ד בג' ימנהו ג' במנין מה שבה"ד מן האחדים |
|
ושטח ד"ב בג'[20] ימנהו ג' במנין מה שבד"ב מן האחדים |
|
הנה אם כן אלו השטחים מקובצים ימנם ג' במנין מה שבא"ה ה"ד ד"ב מן האחדים |
|
אבל מנין מה שבא"ה ה"ד ד"ב מן האחדים הוא מנין מה שבא"ב מן האחדים[21] |
|
אם כן אלו השטחים כלם ימנם ג' במנין מה שבא"ב מן האחדים |
|
אבל שטח א"ב בג' ימנהו ג' במנין מה שבא"ב מן האחדים |
|
אם כן שטח א"ב בג' שוה לאלו השטחים מקובצים |
|
והוא מה שרצינו לבאר |
|
ג כאשר היו שני מספרים מונחים וחולק כל אחד מהם לחלקים כמה שיהיו הנה שטח המספר האחד באחר שוה לשטח [22]חלקי המספר האחד בכל אחד מחלקי המספר האחר |
|
ויהיו המספרים המונחים מספרי א"ב ג"ד |
|
וחולק מספר א"ב לחלקים א"ה ה"ב |
|
וחולק מספר ג"ד לחלקים ג"ז ז"ח ח"ד |
|
ואומר ששטחי א"ה בכל אחד ממספרי ג"ז ז"ח ח"ד עם שטחי ה"ב בכל אחד ממספרי ג"ז ז"ח ח"ד שוים לשטח א"ב בג"ד |
|
| |
|
המופת ששטחי א"ה בחלקי ג"ז ז"ח ח"ד שוים לשטח א"ה בג"ד |
|
וכזה[23] התבאר ששטחי ה"ב בחלקי ג"ז ז"ח ח"ד שוים לשטח ה"ב בג"ד[24] |
|
ואולם שטחי א"ה בג"ד וה"ב בג"ד שוים לשטח א"ב בג"ד |
|
אם כן שטחי חלקי מספר א"ב בכל אחד מחלקי מספר ג"ד שוים לשטח א"ב בג"ד |
|
והוא מה שרצינו לבאר |
|
ד כאשר חולק מספר מה בשני חלקים הנה שטח כל המספר באחד מחלקיו שוה לשטח החלק האחד באחר ולמרובע החלק אשר זכרנו |
|
ויתחלק מספר א"ב בשני חלקים ויהיו חלקים א"ג ג"ב |
|
ואומר ששטח א"ב בג"ב שוה לשטח א"ג בג"ב ולמרובע ב"ג |
|
המופת ששטח א"ג בג"ב עם שטח ג"ב בג"ב שהוא מרובע ג"ב שוה לשטח א"ב בג"ב |
|
| |
|
אם כן שטח א"ב בג"ב שוה לשטח א"ג בג"ב ולמרובע ג"ב |
|
והוא מה שרצינו |
|
ה כאשר חולק מספר מה לחציין והוסף עליו מספר מה הנה שטח התוספת במספר כלו עם התוספת עם מרובע חצי המספר שוה למרובע חצי המספר והתוספת מקובצים |
|
ויחולק מספר א"ב לחציין ויהיו חלקיו א"ב ג"ב |
|
והוסף עליו מספר ב"ד |
|
ואומר ששטח א"ד בד"ב עם מרובע ג"ב שוה למרובע ג"ד |
|
המופת ששטח א"ד בב"ד שוה לשטח ג"ד בב"ד ולשטח א"ג בב"ד שהוא שוה לשטח ב"ג בב"ד |
|
| |
|
וכאשר חובר עמו מרובע ג"ב היה המקובץ שוה לשטח ג"ד בב"ד ולשטח ב"ג בב"ד ולמרובע ג"ב |
|
| |
|
וגם כן הנה שטח ג"ד בג"ד שוה לשטח ג"ד בב"ד ולשטח ג"ד בג"ב |
|
אבל שטח ג"ד בג"ב שוה לשטח ג"ב בב"ד ולמרובע ב"ג |
|
אם כן מרובע ג"ד שוה לשטח ג"ד בב"ד ולשטח ג"ב בב"ד ולמרובע ב"ג וזה שוה לפי מה שבארנו לשטח א"ד[25] בב"ד [26]ולמרובע ג"ב |
|
| |
|
והוא מה שרצינו |
|
ו כאשר נוסף על מספר מונח מספר מה הנה מרובע שני המספרים מחוברים שוה למרובעי המספרים ההם ולכפל שטח זה בזה |
|
ויהיה המספר מספר א"ב ונוסף עליו מספר ב"ג |
|
הנה אומר שמרובע א"ג שוה למרובעי א"ב וב"ג ולכפל שטח א"ב בב"ג |
|
המופת ששטח א"ג בא"ג שוה לשטח א"ב בא"ג ולשטח ב"ג בא"ג |
|
ואולם שטח ב"ג בא"ג שוה לשטח א"ב בב"ג ולמרובע ב"ג[27] |
|
ואולם שטח ב"א בא"ג שוה לשטח א"ב בב"ג ולמרובע א"ב[28] |
|
יהיה אם כן מרובע א"ג שוה לשני מרובעי א"ב ב"ג ולכפל שטח א"ב בב"ג |
|
והוא מה שרצינו |
|
ז כאשר נוסף על מספר מה מספר מה הנה מרובע שני המספרים מחוברים שוה לשטח שני המספרים מחוברים באחד מהם ולשטח זה בזה ולמרובע החלק הנשאר |
|
ויהיה המספר מספר א"ב ונוסף עליו מספר ב"ג |
|
ואומר שמרובע א"ג שוה לשטח א"ג בא"ב ולשטח[29] א"ב בב"ג ולמרובע ב"ג |
|
המופת ששטח א"ג בא"ג שוה לשטח א"ב בא"ג ולשטח ב"ג בא"ג |
|
אבל שטח ב"ג בא"ג שוה לשטח א"ב בב"ג ולמרובע ב"ג |
|
אם כן מרובע א"ג שוה לשטח א"ב בא"ג ולשטח א"ב בב"ג ולמרובע ב"ג[30] |
|
והוא מה שרצינו |
|
ח השטח ההוה מחצי המספר המונח בעצמו שוה לשטח ההוה מחלק מה מהמספר ההוא בחלק השני ולמרובע יתרון אחד מן החלקים על חצי המספר המונח |
|
| |
|
ויהיה המספר המונח מספר א"ב וחולק לחציין בנקודת ג' וחולק איך שקרה בנקודת ד' |
|
ואומר שמרובע מספר א"ג שוה לשטח ההווה ממספר א"ד במספר ד"ב ולמרובע ההוה ממספר ג"ד |
|
המופת שמרובע א"ג שוה לשטח א"ג בג"ד ולשטח א"ג בד"ב מקובצים |
|
אבל שטח א"ד בד"ב שוה לשטח א"ג בד"ב ולשטח ג"ד בד"ב |
|
ונחסר שטח א"ג בד"ב המשותף והיה הנשאר למרובע א"ג שוה לשטח[31] א"ג בג"ד שהוא שוה לשטח ג"ב בג"ד |
|
והנשאר לשטח א"ד בד"ב הוא שטח ג"ד בד"ב |
|
והנה יתרון שטח ג"ב בג"ד על שטח ג"ד בד"ב הוא כמו מרובע ג"ד |
|
אם כן מרובע א"ג שוה לשטח א"ד בד"ב ולמרובע ג"ד |
|
והוא מה שרצינו לבאר |
|
ט כאשר הוכה מספר אחד על מספר מורכב [32]משני מספרים מונחים והיה העולה מספר מה הנה אם הוכה המספר המורכב משני מספרים איזהו שיהיו מאלו השלשה על השלשה יהיה המספר ההוא בעינו |
|
ויוכה מספר א' על שטח ב' בג' ויהיה העולה מספר ד"ה |
|
ואומר שאם הוכה מספר ב' על שטח א' בג' יהיה גם כן העולה מספר ד"ה |
|
המופת שמספר ד"ה ימנהו שטח ב' בג' בשעור אחדי א' |
|
הנה נחלק ד"ה על דמיוני שטח ב' בג' ויהיו חלקיו השוים לשטח ב' בג' חלקי ד"ז ז"ח ח"ה |
|
והנה מספר אלו החלקים הוא כמספר מה שבא' מן האחדים |
|
והוא מבואר שכל אחד מחלקי ד"ז ז"ח ח"ה ימנהו ב' בשעור אחדי ג' לפי שכל אחד מהם שוה לשטח ב' בג' |
|
הנה ד"ה כלו ימנהו ב' במספר מה שימנה כל חלקיו יחד |
|
אבל כל חלקיו יחד ימנם במספרם מוכה על ג' ומספרם הוא כמספר אחדי א' שטח |
|
אם כן ד"ה כלו ימנהו ב' במספר שטח א' בג' |
|
אם כן כבר יוכה מספר ב' בשטח א' בג' ויהיה ד"ה |
|
והוא מה שרצינו |
|
ובזה יתבאר שאיזה מספר[33] שיוכה מאלו השלשה על השטח ההווה מהאחד המספרים הנשארים בשני היה עולה ד"ה ולזה גם כן ימנה ד"ה איזה שיהיה מאלו המספרים במספר שטח אחד מהנשארים בשני |
|
והוא מה שרצינו |
|
י כאשר הוכה מספר אחד על מספר מורכב משלשה מספרים מונחים והיה העולה מספר מה הנה אם הוכה איזה מספר שיהיה מאלו על המספר המורכב מהשלשה הנשארים יהיה המספר ההוא בעינו |
|
ויוכה מספר א' על המספר המורכב ממספרי ג'ד'ה' ויהיה ז"ח |
|
הנה אומר שאם הוכה מספר ד' על המספר המורכב ממספרי א'ג'ה' יהיה העולה ז"ח גם כן |
|
המופת שאנחנו נחלק ז"ח בדמיוני המספר המורכב ממספרי[34] ג'ד'ה' ויהיו חלקיו ז"ט ט"ל ל"ח |
|
הנה מספר אלו החלקים הוא כמספר אחדי א' |
|
מפני שז"ח ימנהו המספר המורכב ממספרי ג'ד'ה' כמספר אחדי א' |
|
וכל אחד מחלקי ז"ט ט"ל ח"ל ימנהו ד' בשעור שטח ג' בה' וזה מבואר ממה שקדם |
|
הנה ז"ח כלו ימנהו ד' במספר מה שימנהו כל חלקיו יחד |
|
אבל כל חלקיו יחד [35]ימנם[36] ד' בשיעור שטח ג' בה' מוכה על מספרם שהוא מספר א' |
|
הנה אם כן ז"ח כלו ימנהו ד' בשעור שטח ג' בה' מוכה על א' |
|
אם כן ז"ח כלו ימנהו ד' כשעור המספר המורכב ממספרי א'ג'ה' |
|
הנה ד' כשהוכה על המספר המורכב ממספרי[37] א'ג'ה' הוא ז"ח גם כן |
|
וכזה התבאר שאיזה שיהיה מאלו המספרים שיוכה על המורכב מהמספרים הנשארים יהיה העולה ז"ח |
|
ובזאת ההדרגה יתבאר לבלתי תכלית רצוני שאם הוכה מספר מה על מספר מורכב מארבעה מספרים והיה מספר מה הנה אם הוכה איזה מספר שיהיה מהם על המספר המורכב מהמספרים הנשארים יהיה העולה המספר ההוא בעינו וכן לאין תכלית |
|
ומפני זה ימנה המספר העולה מהכאת המספר האחד במספר מורכב מהנשארים איזה שיהיה מהמספרים ההם בשעור המספר המורכב מהמספרים הנשארים |
|
והוא מה שרצינו לבאר |
|
י"א כאשר הוכה מספר מה על מספר מורכב משלשה מספרים והיה העולה מספר מה הנה אם הוכה המספר המורכב משני מספרים מהם על המספר המורכב מהמספרים הנשארים יהיה העולה המספר ההוא בעינו |
|
ויוכה מספר א' על המספר המורכב ממספרי ג'ד'ה' והיה ז'ח' |
|
ואומר שאם הוכה שטח א' בד' על שטח ג' בה' יהיה העולה ז'ח' גם כן |
|
המופת שאנחנו נחלוק ז'ח' בדמיוני המספר המורכב ממספרי ג'ד'ה' ויהיו חלקיו ז'ט' ט'ל' ח'ל' |
|
הנה מספר חלקיו הוא כמספר מה שבא' מן האחדים |
|
וכל אחד מאלו החלקים ימנהו שטח ג' בה' בשעור מה שבד' מן האחדים[38] |
|
הנה ז'ח' כלו ימנהו שטח ג' בה' בשעור מה שימנה כל חלקיו יחד |
|
אבל כל חלקיו יחד ימנם כמספרם מוכה על ד' והיה מספרם הוא כמספר אחדי א' |
|
אם כן ז'ח' כלו ימנהו שטח ג' בה' כשעור שטח א' בד' |
|
אם כן כבר יוכה שטח א' בד' על שטח ג' בה' ויהיה העולה ז'ח' |
|
וכזה התבאר שכאשר הוכה המספר המורכב משנים מאלו המספרים איזה שיהיו על המספר המורכב מהשנים הנשארים יהיה העולה ז'ח' גם כן |
|
ובזה הבאור [39]בעינו התבאר שאם הוכה מספר מה על המספר המורכב מארבעה[40] מספרים והיה מספר מה הנה אם הוכה המספר המורכב מהשנים מהם איזה שיהיו על המורכב מהשלשה הנשארים יהיה העולה המספר ההוא בעינו |
|
וכזה התבאר לאין תכלית בכמו זה הבאור בעינו |
|
ומפני זה ימנה המספר העולה המספר[41] המורכב משני מספרים איזה שיהיו מהמספרים ההם כשעור מה שבמספר המורכב מהמספרים הנשארים מן האחדים |
|
והוא מה שרצינו לבאר |
|
י"ב כאשר הוכה מספר מה על המורכב ממספרים כמה שיהיו והיה מספר מה הנה אם הוכה המורכב מאיזה שיהיו מהמספרים ההם על המורכב מהמספרים הנשארים יהיה העולה כמספר ההוא בעינו |
|
ויוכה מספר א' על המורכב ממספרי ב'ג'ד'ה'ז'ח' והיה העולה ט'כ' |
|
ואומ' שאם הוכה המורכב ממספרי ב'ג'ה'ז'[42] על המורכב ממספרי א'ד'ח'[43] יהיה העולה ט'כ' גם כן |
|
המופת שמספר ט'כ'[44] ימנהו המספר המורכב ממספרי ב'ג' כשעור אחדי המספר המורכב ממספרי א'ד'ה'ז'ח'[45] |
|
הנה נחלק ט'כ' בדמיוני א'ד'ה'ז'ח'[46] ויהיו חלקיו ט'ל' ל'מ' מ'ס' ס'כ' |
|
הנה מספר אלו החלקים כשעור אחדי שטח ב' בג' |
|
וגם כן הנה כל אחד מאלו החלקים ימנהו מורכב א'ד'ח'[47] כשעור אחדי שטח ה'[48] בז' לפי שכל אחד מהם שוה למורכב א'ד'ה'ז'ח' |
|
| |
|
והנה ט'כ' כלו ימנהו מורכב א'ד'ח'[49] כשעור מה שימנה כל חלקיו יחד |
|
| |
|
אבל חלקיו יחד ימנם מורכב א'ד'ח' בשעור שטח ה'[50] בז' מוכה על מספרם שהוא כמספר שהוא שטח ב' בג' והעולה כבר התבאר שהוא המספר המורכב ממספרי ב'ג'ה'ז'[51] |
|
| |
|
אם כן ט'כ' כלו ימנהו המספר[52] המורכב ממספרי א'ד'ח'[53] כמספר שטח ה' בז' מוכה על שטח ב' בג' והעולה כבר התבאר שהוא המספר המורכב ממספרי ב'ג'ה'ז' |
|
| |
|
אם כן ט'כ' כלו ימנהו המורכב ממספרי א'ד'ח' כשעור אחדי המספר המורכב ממספרי ב'ג'ה'ז' |
|
אם כן כבר יוכה מורכב א'ד'ח' במורכב ב'ג'ה'ז' ויהיה העולה מספר ט'כ'[54] |
|
וכזה התבאר שאם הוכה מורכב איזה שיהיו מאלו [55]המספרים על המורכב מהמספרים הנשארים יהיה העולה ט'כ' |
|
ובזה התבאר באיזה מספר מורכב מכמה מספרים שיהיו שאם הוכה המספר המורכב ממספרים מה מהם על המספר המורכב מהמספרים הנשארים יהיה[56] העולה המספר ההוא בעינו |
|
ולזה ימנה המספר העולה המספר המורכב מאיזה[57] שיהיו מהמספרים ההם כמספר אחדי המורכב מהמספרים הנשארים |
|
והוא מה שרצינו |
|
י"ג המספר המורכב ממספרים מה יחסו אל המספר המורכב ממספרים אחרים מספרם כמספר המספרים הקודמים כמו היחס המחובר מהמספרים הקודמים אל המספרים הנמשכים |
|
ויהיה מספר א' מורכב ממספרי ב'ג'ד'ה'ז' |
|
ומספר ח' מורכב ממספר ט'כ'ל'מ'נ' |
|
ואומר שיחס א' אל ח' מחובר מחמשה יחסים מיחס ב' אל ט' ומיחס ג' אל כ' ומיחס ד' אל ל' ומיחס ה' אל מ' ומיחס ז' אל נ' |
|
המופת שאנחנו נכה המורכב ממספר ג'ד'ה'ז' במספר ט' ונשים העולה ס' |
|
הנה מורכב ג'ד'ה'ז' הוכה בב' והיה א' |
|
והוכה בט' ויהיה[58] ס' |
|
הנה אם כן יחס א' אל ס' כיחס ב' אל ט' |
|
וגם כן הנה נכה מורכב ט'ד'ה'ז' בכ' ונשים העולה מספר ע' |
|
הנה מורכב ט'ד'ה'ז' הוכה בג' והיה ס'[59] |
|
והוכה בכ' והיה ע' |
|
הנה יחס ס' אל ע' כיחס ג' אל כ' |
|
וגם כן הנה נכה מורכב ט'כ'ה'ז' בל' ונשים העולה[60] מספר פ'[61] |
|
ויתבאר כמו הבאור הקודם שיחס ע' אל פ' הוא כיחס ד' אל ל'[62] |
|
וגם כן הנה נכה מורכב ט'כ'ל'ז' במספר מ' והיה צ' |
|
ויתבאר גם כן שיחס פ' אל צ' הוא כיחס ה'[63] אל מ' |
|
וכזה התבאר שיחס צ' אל ח'[64] כיחס ז'[65] אל נ' |
|
ובהיות הענין כן הוא מבואר שיחס א'[66] אל ח' מחובר מחמשה יחסים מיחס א' אל ס' ומיחס ס'[67] אל ע' ומיחס ע' אל פ' ומיחס פ' אל צ' ומיחס צ' אל ח' |
|
וכבר התבאר שכל יחס מאלו היחסים הוא כמו גילו מיחסי מספרי ב'ג'ד'ה'ז' אל מספרי ט'כ'ל'נ'מ' |
|
אם כן יחס א' אל ח' מחובר מחמשה יחסים מיחס ב' אל ט' ומיחס ג' אל כ' ומיחס ד' אל ל' ומיחס ה' אל מ' ומיחס ז' אל נ' |
|
והוא מה שרצינו לבאר |
|
י"ד היחס המחובר ממספרים [68]מה קודמים אל מספרים מה נמשכים הנה כאשר הומר סדור המספרים הגיליים ונשארו הקודמים קודמים והנמשכים נמשכים ישאר היחס המחובר כמו היחס המחובר הראשון |
|
ויהיו המספרים הקודמים מספרי א'ב'ג'ד' |
|
והמספרים הנמשכים מספרי ה'ז'ח'ט' |
|
והיה היחס המחובר מיחס א' אל ה' ומיחס ב' אל ז' ומיחס ג' אל ח' ומיחס ד' אל ט' כיחס כ' אל ל' |
|
ואומר שאם הומר סדור הגיליים ולוקח היחס המחובר מיחס א' אל ה'[69] ומיחס ב' אל ח'[70] ומיחס ג' אל ט' ומיחס ד' אל ז' יהיה גם כן כיחס כ' אל ל' |
|
המופת שאנחנו נשים המספר המורכב ממספרי א'ב'ג'ד'[71] מספר מ' והמספר המורכב ממספרי ה'ז'ח'ט' מספר משלפניה נ' |
|
הנה יחס מ' אל נ' הוא כמו היחס המחובר ממספרי א'ב'ג'ד' אל מספרי ה'ז'ח'ט' |
|
ומספר המורכב ממספרי ה'ז'ח'ט' הוא כמו המספר המורכב ממספרי ח'ה'ט'ז' |
|
אם כן יחס מ' אל נ' הוא כמו היחס המחובר ממספרי א'ב'ג'ד' אל מספרי ח'ה'ט'ז' |
|
וכבר היה יחס מ' אל נ' כמו היחס המחובר ממספרי א'ב'ג'ד' אל מספרי ה'ז'ח'ט' |
|
| |
|
א"כ (מפתיחת אקלידיס) היחס המחובר ממספרי א'ב'ג'ד' אל מספרי ה'ז'ח'ט' הוא כמו היחס[72] המחובר ממספרי א'ב'ג'ד' אל מספרי ה'ז'ח'ט' כיחס כ' אל ל' |
|
אם כן היחס המחובר ממספרי א'ב'ג'ד' אל מספרי ח'ה'ט'ז' הוא כיחס כ' אל ל' גם כן |
|
והוא מה שרצינו לבאר |
|
ובזה התבאר שאם הומר סדר הקודמים ונשארו הקודמים קודמים שהיחס המחובר ישאר אחד בעינו[73] |
|
והוא מה שרצינו |
|
ט"ו כל מספר שיהיה ראשון אצל המורכב ממספרים מה מונחים הנה הוא ראשון אצל כל אחד מהם |
|
ויהיה מספר א' ראשון אצל מספר ה' ויהיה מספר ה' מורכב ממספרי ב'ג'ד' |
|
ואומר שמספר א' ראשון אצל כל אחד ממספרי ב'ג'ד' |
|
המופת שאי אפשר זולת זה |
|
שאם היה אפשר הנה יהיו מספרי א'ג' משותפים וימנם בהכרח מספר מה ונניחהו מספר ז' |
|
אבל ג' ימנה ה' וזה שהוא ימנהו כמספר מה שבמורכב ב'ד'[74] מן האחדים |
|
הנה ז' ימנה ה' |
|
וכבר היה מונה א' |
|
אם כן יהיו א'ה' משותפים |
|
אבל כבר הונח א' ראשון אצל מספר ה' זה שקר |
|
אם כן מספר א' ראשון אצל כל אחד ממספרי ב'ג'ד' |
|
והוא מה שרצינו לבאר |
|
י"ו כל מספר שיהיה ראשון אצל כל המספרים הראשונים הקטנים משרש המרובע המוסיף עליו היותר קרוב לו הנה הוא ראשון |
|
ויהיה מספר א' ראשון [75]אצל כל המספרים הקטנים משרש מספר ב' והוא המרובע היותר קרוב למספר א' המוסיף עליו |
|
ויהיה יסוד מספר ב' מספר ג' |
|
ויהיו המספרים הראשונים הקטנים ממספר ג' מספרי ד'ה'ז' |
|
ויהיה א' ראשון אצל כל אחד מהם |
|
ואומר שמספר א' הוא ראשון |
|
המופת שאם היה[76] אפשר זולת זה ימנהו מספר מה והוא ח' וימנהו כמספר אחדי ט' |
|
והוא מבואר שאין כל אחד ממספרי ח'ט' בלתי קטן מג' |
|
שאם היה אפשר זה לא יהיה שטח ח' בט' והוא א' קטן משטח ג' בג' והוא ב' |
|
וכבר הונח א' קטן מב' זה שקר |
|
הנה אם כן אחד ממספרי ח'ט' קטן מג' |
|
ויהיה הקטן מג' מספר ח' |
|
הנה מספר ח'[77] אם שיהיה ראשון ואם שיהיה מורכב |
|
ואם היה ראשון והוא קטן מג' יהיה א' בלתי ראשון אצל כל הראשונים הקטנים מג' וכבר הונח ראשון אצל כלם זה שקר |
|
ואם היה מורכב הנה ימנהו בהכרח מספר ראשון קטן[78] ממספר ח' ולזה יהיה קטן מג' ויתחייב השקר הקודם בעינו |
|
אם כן לא ימנה שום מספר מספר א' ולזה יהיה מספר א' מספר ראשון |
|
וזה הוא מה שרצינו |
|
י"ז כאשר לוקח ממספר[79] מונח חלק מה מונח או חלקים מונחים ולוקח[80] עוד מהנשאר חלק אחר[81] מונח או חלקים אחרים[82] מונחים[83] וכן בזה הדרך מה שהגיע הנה אם הומר הסדור יהיה הנשאר באחרונה אחד בעינו ומקובץ החלקים אחד בעינו |
|
ויהיה המספר המונח מספר א' |
|
והחלקים הם הנקראים במספרי ב'ג'ד' והם חלק מב' במספר א' וה'[84] חלקים מג' בנשאר וז' חלקים[85] מד' בנשאר |
|
ואומר שחלק אחד מב' מא'[86] עם ה' חלקים מג' בנשאר ועם ז' חלקים מד' בנשאר הנה כשהתקבץ זה כלו יהיה שוה לז' חלקים מד' במספר א' וחלק אחד מב' בנשאר וה' חלקים מג' בנשאר |
|
המופת שאנחנו נשים מספר ח' פחות אחד מספר ב' |
|
ונשים מספרי ח'ט'[87] שוים לג' |
|
ומספרים ז'כ' שוים לד' |
|
ויהיה חלק מב' מא' מספר פ' |
|
וישאר מספר ל' |
|
ויהיו ה'[88] חלקים מג'[89] במספר ל' מספר מ' |
|
וישאר מספר נ' |
|
ויהיו ז' חלקים מד' במספר [90]נ'[91] מספר ס' |
|
וישאר מספר ע' |
|
וגם כן הנה ז'[92] חלקים מד' במספר א' מספר צ' |
|
ויהיה הנשאר מספר ק' |
|
ויהיה חלק מב' ממספר ק' מספר[93] ר' |
|
ויהיה הנשאר מספר ש' |
|
ויהיו ה'[94] חלקים מג' במספר ש' מספר ת' |
|
ויהיה הנשאר מספר ץ' |
|
ואומר שמספרי[95] ע'ץ' שוים |
|
המופת בו מפני שהמספר פ' אחד מב' במספר א' |
|
יהיה במספר א' מדמיוני[96] פ' כמו מה שבמספר ב' מן האחדים |
|
ולזה יהיה במספר ל' מדמיוני פ' כמו מה שבמספר ב' מן האחדים[97] פחות אחד |
|
אבל ח' הוא פחות אחד מב' |
|
אם כן במספר ל' מדמיוני פ' כמו מה שבמספר ח'[98] מן האחדים |
|
אם כן יחס א' אל ל' כיחס ב' אל ח' |
|
לפי שמספרי ב'ח' הוכו במספר פ' ויהיו מספרי א'ל' |
|
וגם כן הנה נשים חלק מג' במספר ל' מספר ף' |
|
ולזה יהיה במספר מ'[99] מדמיוני ף'[100] כמו מה שבמספר ה' מן האחדים |
|
ולזה יהיה גם כן במספר נ' מדמיוני ף' כמו מה שבמספר ט'[101] מן האחדים |
|
ויתבאר על האופן הקודם שיחס ל' אל נ' כיחס ג'[102] אל ט' |
|
וכזה התבאר שיחס נ' אל ע' הוא כיחס ד' אל כ' |
|
אם כן יחס א' אל ע' מחובר ממספרי ב'ג'ד' אל מספרי ח'ט'כ' |
|
וכזה יתבאר שיחס א' אל מספר ץ' מחובר מיחס מספרי[103] ד'ב'ג' אל מספרי כ'ח'ט' |
|
אבל היחס המחובר ממספרי ב'ג'ד' אל מספרי ח'ט'כ' שוה אל היחס המחובר ממספרי ד'ב'ג' אל מספרי כ'ח'ט' |
|
אם כן יחס א' אל ע' ואל ץ' אחד |
|
ולזה יהיה ע' כמו ץ' |
|
ולזה גם כן יחויב שיהיו מספרי פ'מ'ס' מקובצים שוים למספרי צ'ר'ת' מקובצים |
|
וזה שיתרון א'[104] על ע' הוא מספרי פ'מ'ס' |
|
ויתרון א' על ץ'[105] הם מספרי צ'ר'ת'[106] |
|
וכבר התבאר שמספר ע' שוה למספר ץ'[107] |
|
אם כן מספרי פ'מ'ס' שוים למספרי צ'ר'ת'[108] |
|
והוא מה שרצינו לבאר |
|
י"ח כאשר הוכה מספר מונח במספר מה מונח ולוקח מהעולה מההכאה[109] חלק מה מונח או חלקים מה[110] מונחים וכן מה שהגיע מלקיחת החלק או החלקים ומההכאות[111] הנה אם הומר הסדור יהיה הנשאר באחרונה אחד בעינו |
|
ויהיה המספר המונח מספר א' |
|
ויוכה במספר [112]ב' |
|
וילקח מהעולה ה'[113] חלקים מג' וילקח מהנשאר ז' חלקים מד' וישאר מספר מה |
|
ואומר שאם הומר הסדור שילקח ממספר א' ז' חלקים מד'[114] ויוכה הנשאר במספר ב'[115] וילקח מהנשאר ה' חלקים מג' הנה ישאר המספר ההוא בעינו שנשאר בסדור האחר |
|
וזה שאנחנו נשים על מספר ב'[116] אחד |
|
ונשים מספרי ה'ט' שוים לג' |
|
ומספרי ז'כ' שוים לד' |
|
ויוכה א' על ב' ויהיה העולה ל' |
|
ויהיו ה' חלקים מג' במספר ל' מספר מ' |
|
וישאר מספר נ' |
|
ויהיו ז' חלקים מד' במספר נ' מספר ס' |
|
וישאר מספר ע' |
|
וגם כן הנה נקח ז' חלקים מד' במספר א' ויהיה מספר פ' |
|
וישאר מספר צ' |
|
ויוכה צ' בב' ויהיה ק' |
|
ויהיו ה'[117] חלקים מג' במספר ק' מספר ר' |
|
וישאר מספר ש' |
|
ואומר שמספרי ש'ע' שוים |
|
המופת כי א' הוכה בב' והיה ל' |
|
הנה יחס א' אל ל' כיחס אחד[118] אל ב' |
|
ויתבאר ממה שקדם בתמונה הקודמת שיחס ל' אל נ' הוא כיחס ג' אל ט' |
|
ויחס נ' אל ע'[119] הוא כיחס ד' אל כ' |
|
הנה אם כן יחס א' אל ע' מחובר ממספרי אחד ג'ד'[120] אל מספרי ב'ט'כ' |
|
ובזה התבאר שיחס א' אל ש'[121] מחובר ממספרי ד' אחד ג' אל מספרי כ'ב'ט'[122] |
|
אבל היחס המחובר ממספרי אחד ג'ד'[123] אל מספרי ב'ט'כ' הוא כמו היחס המחובר ממספרי ד' אחד ג' אל מספרי כ'ב'ט' |
|
אם כן יחס א' אל ע' ואל ש' אחד |
|
אם כן ע' כמו ש' |
|
והוא מה[124] שרצינו לבאר |
| We have called one a number, although it is not a number, as a metaphor, for the proof does not change by that, and this is clarified by the proof in proposition 13 of this section. | והנה קראנו האחד מספר ואם איננו מספר על צד ההעברה[125] כי צד המופת לא יתחלף בזה וזה מבואר מהמופת הנעשה בזה בתמונת י"ג מזה המאמר |
Arithmetic progressions and sums |
|
|
י"ט כל מספר מונח הנה מספר המספרים הנמשכים המתחילים מן האחד עד שהגיע ההמשך אל המספר המונח הוא[126] כמספר מה שבמספר המונח[127] מן האחדים |
|
ויהיה המספר המונח מספר א'ב' |
|
ואומר שמספר המספרים הנמשכים מתחילין מן האחד עד שהגיע ההמשך[128] אל מספר א'ב' הוא[129] כמספר [130]מה שבמספר א'ב' מן האחדים |
|
המופת שאנחנו נחלוק א'ב' בדמיוני מה שיש בו מן האחדים והם א'ג' ג'ד' ד'ה' ה'ב' |
|
הנה א'ג' הוא אחד |
|
וכאשר חובר עמו ג'ד' שהוא אחד היה א'ד' המספר הנמשך לא'ג' לאחריו |
|
וכזה התבאר שמספר א'ה' הוא הנמשך למספר א'ד' לאחריו ושמספר א'ב' הוא המספר הנמשך למספר א'ה' לאחריו |
|
הנה אם כן מספרי א'ג' א'ד' א'ה' א'ב' נמשכים ומתחילים מן האחד ומספרם כמספר מה שבא'ב' מן האחדים |
|
והוא מה שרצינו לבאר |
|
ובזה התבאר מזאת התמונה בעצמה שמספר אחדי האחרון מהמספרים הנמשכים מתחילים מן האחד הוא כמספר המספרים ההם מתחילים מן האחד |
|
והוא מה שרצינו לבאר |
|
כ כל מספר זוג הנה מספר[131] המספרים הנפרדים הנמשכים מן האחד והאחד עמהם עדיו שוה למספר הזוגות הנמשכים עדיו[132] |
|
ויהיה מספר א'ב' מספר זוג[133] |
|
ואומר[134] שמספר הזוגות הנמשכים עד מספר א'ב' שוה למספר המספרים הנפרדים הנמשכים עד מספר א'ב' והאחד עמהם |
|
המופת שנחלק א'ב' במנין מה שבו מן האחדים והם א'ג' ג'ד' ד'ה' ה'ב' |
|
הנה מפני שא'ב' הוא זוג יהיה א'ה' נפרד |
|
לפי שא'ב' מוסיף על א'ה' אחד[135] |
|
ובזה התבאר שא'ד' זוג וא'ג' נפרד והוא האחד |
|
הנה אם כן מספר הזוגות הנמשכים עדיו הם כמו מספר הנפרדים |
|
והוא מה שרצינו |
|
ומזאת התמונה התבאר שכל מספר נפרד מונח יהיה מספר המספרים הנפרדים מתחילים מן האחד הנמשכים עדיו מוסיף על מספר הזוגות אחד |
|
וזה שכאשר נגרע מהם זה המספר הנפרד היה האחרון זוג ויהיה מספר הזוגות שוה למספר המספרים הנפרדים |
|
יהיה אם כן מספר הנפרדים מוסיף אחד על מספר[136] הזוגות |
|
כ"א כאשר נמשכו אחר מספר מונח מספרים מה הנה מספר האחרון שבמספרים ההם[137] מוסיף על המספר המונח מן האחדים כמו מספר המספרים הנמשכים ההם |
|
וימשכו אחר [138]מספר א'ב' המונח מספרי א'ג' א'ד' א'ה' |
|
ויהיה מספר אלו המספרים מספר ז' |
|
ואומר שמספר[139] א'ה' מוסיף על מספר א'ב' מספר ז' |
|
המופת שאנחנו נשים מספר המספרים הנמשכים עד א'ב' מספר ח' |
|
הנה אם כן מספר אחדי א'ב' הם ח' |
|
וגם כן הנה מספר המספרים הנמשכים עד א'ה' מוסיף על מספר המספרים הנמשכים עד א'ב' מספר ז' |
|
הנה אם כן מספר המספרים הנמשכים עד א'ה' הם מספרי ח'ז' מקובצים |
|
אם כן מספר מה שבא'ה' מן האחדים הוא כמו מספרי ח'ז' מקובצים |
|
ואולם מספר אחדי א'ב' הוא ח' |
|
אם כן מספר א'ה' מוסיף על א'ב' כמו מספר ז' |
|
והוא מה שרצינו לבאר |
|
כ"ב כאשר היה מספר הנמשכים לפני מספר מונח כמו מספר הנמשכים לאחריו הנה יתרון המספר המונח על הראשון מהנמשכים לו לפניו[140] הוא כמו יתרון האחרון מהנמשכים לו[141] לאחריו על המספר המונח |
|
ויהיה המספר המונח מספר א'ב' |
|
ויהיו המספרים הנמשכים לפניו מספרי א'ג' א'ד' א'ה' |
|
והמספרים הנמשכים לו לאחריו מספרי א'ז' א'ח' א'ט' |
|
ואומר שיתרון מספר א'ב' על[142] מספר א'ה' שוה ליתרון מספר[143] א'ט' על מספר א'ב' |
|
המופת שמספר מספרי א'ה' א'ד' א'ג' שוה[144] למספר מספרי א'ז' א'ח' א'ט' |
|
אבל מספר מספרי א'ד' א'ג' א'ב' שוה למספר מספרי א'ה' א'ד' א'ג' |
|
יהיה אם כן מספר מספרי א'ד' א'ג' א'ב' שוה למספר מספרי א'ז' א'ח' א'ט' |
|
ויתרון א'ב' על א'ה' הוא כמספר מספרי א'ד' א'ג' א'ב' |
|
ויתרון א'ט' על א'ב' הוא כמספר מספרי א'ז' א'ח' א'ט' |
|
אם כן יתרון א'ב' על א'ה' שוה ליתרון א'ט' על א'ב' |
|
והוא מש"ל |
|
כ"ג כאשר היה מספר המספרים הנמשכים לפני מספר מונח כמו מספר הנמשכים לאחריו הנה אם היה הראשון מהנמשכים לפניו זוג הנה האחרון מהנמשכים לאחריו זוג ואם נפרד נפרד |
|
ויהיה המספר המונח מספר ד' |
|
והנמשכים לו לפניו מספרי ג'ב'א' |
|
והנמשכים לו לאחריו מספרי ה'ז'ח' |
|
ואומר שאם היה מספר א' זוג שמספר ח' זוג ואם היה מספר א' נפרד היה [145]מספר ח' נפרד |
|
המופת שאנחנו נשים יתרון מספר ד' על מספר א' מספר ט' |
|
ולזה היה יתרון מספר ח' על מספר ד' מספר ט' |
|
הנה אם כן יתרון מספר ח' על מספר א' הוא כמו שני דמיוני מספר ט' |
|
אבל שני דמיוני מספר ט' הוא זוג הנה אם כן יתרון מספר ח' על מספר א' הוא זוג |
|
ולזה אם יהיה א'[146] זוג יהיה ח' זוג |
|
ואם היה א' נפרד יהיה ח' נפרד |
|
והוא מה שרצינו לבאר |
|
כ"ד כאשר חוברו שני מספרים והיה יתרון מספר מה מהם על אחד כמו חסרון המספר השני ממספר מה מונח הנה שני המספרים מחוברים שוים אל המספר הנמשך אל המספר המונח לאחריו |
|
ויהיה תוספת א' על אחד כמו חסרון מספר ב' ממספר ג' המונח |
|
ויהיה המספר הנמשך אל ג' לאחריו מספר ד'ה' |
|
ואומר שמספרי א'ב' מחוברים שוים למספר ד'ה' |
|
המופת שנגרע מד'ה' אחד והוא ה'ז' וישאר ד'ז' [147]שוה לג' |
|
ונשים חסרון ב' מג' מספר ז'ח' |
|
וישאר ד'ח' שוה לב' |
|
אבל ח'ז' הוא גם כן תוספת א' על אחד |
|
וז'ה' הוא אחד |
|
אם כן יהיה ח'ה' שוה לא' |
|
וכבר היה ד'ח' שוה לב' |
|
אם כן ד'ה' שוה לב'א' מחוברים |
|
והוא מה שרצינו |
|
כ"ה כאשר חוברו שני מספרים והיה תוספת אחד מהם על מספר מונח שוה לחסרון האחר מהמספר המונח הנה שניהם מחוברים שוים לכפל המספר המונח |
|
ויהיה חסרון מספר א' ממספר ב' המונח שוה לתוספת ג'ה' על מספר ב' המונח |
|
ואומר שא' וג'ה' נחברים שוים לכפל מספר ב' המונח |
|
המופת שנבדיל מג'ה' מה שהוסיף על ב' המונח והוא ה'ז' |
|
וישאר ג'ז' שוה לב' |
|
וגם כן הנה מפני שה'ז' הוא חסרון מספר א' ממספר ב' כבר יחובר ה'ז' עם א' ויהיה כמו ב' |
|
וכבר היה ג'ז' שוה לב' |
|
אם כן מספרי א'[148] וג'ה' נחברים שוים לשני כפלי מספר ב' |
|
והוא מה שרצינו |
|
כ"ו כאשר נקבצו המספרים הנמשכים בדרך המספר מתחילים מן האחד והיה מספר המספרים [149]שחוברו זוג הנה העולה שוה אל שטח חצי מספר המספרים ההם במספר הנמשך אחר האחרון |
|
ויהיו המספרים הנמשכים א'ב' ג'ד' ה'ו' |
|
ויהיה המספר הנמשך אחר ו' מספר ז' |
|
וא' הוא אחד ונקראהו מספר בכל זאת החקירה על צד ההעברה |
|
ואומר שא'ב' ג'ד' ה'ו' מקובצים שוה אל הנערך מחצי מספרם על מספר ז' |
|
המופת כי מפני שא' הוא אחד יהיו ו' וא' מקובצים שוים לז' |
|
אבל תוספת ב' על אחד שוה לחסרון ה' ממספר ו' מפני שהתוספת הוא אחד |
|
אם כן ב'ה' נחברים שוה לז' |
|
אבל תוספת ג' על אחד שוה לחסרון ד' מו'[150] לפי שהתוספת הוא שנים |
|
אם כן ג'ד' מחוברים שוים לז' |
|
אם כן נקבץ מספרי א'ב' ג'ד' ה'ו' ימנהו ז' כשעור חצי מספרם לפי שכל שנים מהם ימנוהו פעם אחת |
|
והוא מה שרצינו |
|
והוא מבואר שבזה הבאור בעינו יתבאר לאין תכלית ואין ספק שהוא מחוייב שנגיע בזאת ההדרגה באחרונה אל שני מספרים נמשכים כמו ג'ד' במשלנו זה |
|
שאם היה אפשר זולת זה יהיה ביניהם באחרונה מספר אחר אם כן המספר הגדול מהם מוסיף על גילו שנים |
|
ונשים חסרון הגדול מהם ממספר האחרון מספר ט' |
|
ולזה יהיה יתרון הקטן מאלו שני המספרים הגיליים על האחד מספר ט' |
|
וכבר היה יתרון הגדול על הקטן שנים |
|
יהיה א"כ יתרון הגדול על האחד מספר ט'[151] נחבר עם שנים |
|
וכבר היה יתרון האחרון על הגדול מספר ט' |
|
יהיה אם כן יתרון האחרון על האחד כמו שני דמיוני מספר ט' מקובצים עם שנים |
|
אבל שני דמיוני מספר ט' מקובצים עם שנים הוא זוג |
|
יהיה אם כן יתרון האחרון על האחד[152] מספר[153] זוג |
|
אם כן האחרון נפרד וכבר היה זוג זה שקר |
|
אם כן הוא מחוייב שיגיע באחרונה אל שני מספרים נמשכים ובזה התאמת הספור |
|
כ"ז כאשר חוברו המספרים הנמשכים בדרך המספר והאחד[154] עמהם והיה מספר המספרים שחוברו נפרד הנה העולה שוה אל שטח המספר האמצעי מהם במספר האחרון |
|
ויהיו המספרים הנמשכים א'ב' ג'ד' ה'ו' ז' וא' הוא אחד |
|
ואומר שמספרי א'ב' ג'ד' ה'ו' ז' נחברים שוים אל שטח ד' בז' |
|
המופת שחסרון ג' מד' שוה לתוספת ה' על ד' |
|
[155]אם כן ג'ה' מקובצים שוים לשני כפלי ד' |
|
וגם כן חסרון ב' מד' שוה לתוספת ו' על ד' |
|
אם כן ב'ו' נחברים שוים לשני כפלי ד' |
|
ובזה התבאר שא'ז' נחברים שוים לשני כפלי ד' |
|
אם כן נקבץ מספרי א'ב' ג'ד' ה'ו'ז' ימנהו ד' כמספר המספרים ההם |
|
לפי שכל שנים מהם ימנם ד' שני פעמים וד' ימנה עצמו פעם אחת |
|
אם כן נקבץ מספרי א'ב' ג'ד' ה'ו'ז' ימנהו ד' כמספר המספרים ההם |
|
אבל מספר המספרים ההם הם כמו מספר ז' |
|
אם כן נקבץ מספרי א'ב' ג'ד' ה'ו'ז' ימנהו ד' כמספר אחדי ז' |
|
אם כן כבר יוכה ד' בז' ויהיה שוה לנקבץ מספרי א'ב' ג'ד' ה'ו'ז' |
|
והוא מה שרצינו |
|
ואין ספק שבזאת ההדרגה נגיע אל האחרון כהגיענו אל הראשון לפי שמספר ד' הוא אמצעי בין האחרון והראשון ולזה יהיה מספר המספרים הנמשכים לפני האמצעי כמו מספר המספרים הנמשכים לאחריו |
|
כ"ח כאשר היו מספרים נמשכים מתחילים מן האחד והיה מספר המספרים נפרד הנה אם הוכה חצי מספר האחרון במספר הנמשך לו לאחריו יהיה העולה שוה אל נקבץ המספרים ההם |
|
ויהיו המספרים מספרי[156] א'ב' ג'ד' ה'ו'ז' |
|
וא' הוא אחד[157] |
|
ויהיה המספר הנמשך למספר ז' לאחריו מספר ח' |
|
ואומר ששטח חצי מספר ז' במספר ח' שוה אל נקבץ מספרי א'ב' ג'ד' ה'ו'ז' |
|
המופת כי מפני שא' עם ז' נחברים שוים לשני כפלי ד' לפי שא' הוא אחד |
|
וח' שוה לא'ז' מקובצים לפי שא' הוא אחד |
|
יהיה ח' שוה לכפל ד' |
|
וכבר התבאר שנקבץ מספרי א'ב' ג'ד' ה'ו'ז' שוה לשטח ד' בז' ושטח ד' בז'[158] שוה לשטח כפל ד' בחצי מספר ז' |
|
| |
|
לפי שהצלעות מספיקות רצוני לומר שיחס ד' אל כפל ד' כיחס חצי מספר ז' אל ז' |
|
אם כן שטח ד' בז' שוה לשטח ח' בחצי מספר ז' |
|
ואם כן שטח ח' בחצי מספר ז' שוה לנקבץ מספרי א'ב' ג'ד' ה'ו'ז' |
|
והוא מה שרצינו |
|
כ"ט נקבץ הנפרדים הנמשכים בדרך המספר[159] והאחד[160] עמהם שוה למרובע המספר האמצעי בין הנפרד האחרון והאחד |
|
ויהיו המספרים מספרי א'ב' ג'ד' ה'ו' ז'ח'ט' |
|
ומספרי א'ג'ז' ה'ט' הם נפרדים |
|
ואומר [161]שנקבץ נפרדי א'ג' ה'ז'ט' שוה למרובע האמצעי בין ט' ובין א' |
|
המופת שהמספר האמצעי אם שיהיה זוג ואם שיהיה נפרד |
|
ויהיה תחלה נפרד כמו הענין במשלינו זה |
|
ואומר שמספרי א'ג'ז' ה'ט' מקובצים שוים למרובע מספר[162] ה' שהוא האמצעי |
|
המופת שא' יחובר עם ט' ויהיה כמו כפל ה' |
|
וג' יחובר עם ז' ויהיה כמו כפל ה'[163] |
|
אם כן נקבץ מספרי א'ג'ז' ה'ט' ימנהו ה' כשעור מספר הנפרדים |
|
והוא מבואר[164] שלא ישאר נפרד באחד הפאות שלא יתחבר עם גילו בפאה האחרת[165] לפי שמספר המספרים ההם אשר אחר המספר האמצעי הוא כמו מספר המספרים אשר לפניו |
|
והזוגות יתחברו עם הזוגות והנפרדים עם הנפרדים כמו שקדם |
|
אם כן מספר הנפרדים אשר לפני האמצעי כמו מספר הנפרדים אשר לאחריו |
|
אבל מספר הנפרדים אשר לפני ה' הוא כמו חצי מספר ה' כשנגרע ממנו אחד |
|
יהיה אם כן מספר מספרי א'ג' ז'ט' כמו מספר ה' פחות אחד |
|
אם כן נקבץ מספרי א'ג'ז'ט'[166] ימנהו ה' כשעור אחדי ה' פחות אחד |
|
וה' ימנה עצמו פעם אחת |
|
אם כן נקבץ מספרי א'ג'ה' ז'ט' ימנהו ה' בשעור אחדי ה' |
|
אם כן נקבץ מספרי א'ג'ה' ז'ט' שוה למרובע ה' |
|
ויהיה גם כן האמצעי זוג כמו הענין במספרי א'ב' ג'ד' ה'ו' ז' |
|
ואומר שנקבץ מספרי א'ג' ה'ז' שוה[167] למרובע מספר ד' שהוא האמצעי |
|
והנה יתבאר בכמו הבאור הקודם שנקבץ מספרי א'ג' ה'ז' ימנהו ד' כשעור מספר[168] המספרים הנפרדים |
|
ולפי שהיה מספר ד' זוג יהיו הנפרדים שלפניו שוים לחצי מספרו |
|
וכבר התבאר שמספר הנפרדים אשר אחריו שוה למספר הנפרדים לפניו |
|
א"כ מספר הנפרדים לאחריו[169] שוה לחצי מספרו |
|
ולזה יהיה מספר הנפרדים אשר לפניו ולאחריו שוה למספר ד' |
|
וכבר התבאר שנקבץ מספרי א'ג' ה'ז' ימנהו ד' במספר הנפרדים[170] ההם אשר הוא שוה למספר ד' |
|
אם כן נקבץ מספרי א'ג' ה'ז' שוה למרובע ד' |
|
והוא מה שרצינו לבאר |
|
ל כאשר חובר נקבץ הנמשכים בדרך המספר מתחילים מן האחד עד[171] מספר מה מונח עם נקבץ הנמשכים [172]מתחילים מן האחד עד המספר הנמשך אחר המספר המונח הנה העולה שוה למרובע המספר הנמשך אחר המספר המונח |
|
ויחובר נקבץ מספרי א'ב' ג'ד' ה' עם נקבץ מספרי א'ב' ג'ד' ה'ו' |
|
ויהיה א' אחד |
|
ואומר שהעולה שוה למרובע ו' |
|
| |
|
המופת שאנחנו נשים המספר הנמשך אחר ו' מספר ז' |
|
והוא מבואר שנקבץ מספרי א'ב' ג'ד' ה' שוה לשטח חצי מספר ה' בו' |
|
ונקבץ א'ב'ג'ד'ה'ו' שוה לשטח חצי מספר[173] ו' בז' |
|
אבל שטח חצי מספר ו' בז' שוה לשטח חצי מספר ז' בו' מפני שהצלעות מספיקות |
|
אם כן נקבצי א'ב'ג'ד'ה' א'ב'ג'ד'ה'ו' מחוברים שוים לשטח חצי מספר ה' בו' ולשטח חצי מספר ז' בו' והוא כמו שטח חצי מספרי ה'ז' בו' |
|
| |
|
ומפני שה' וז' הוא כמו כפל ו' יהיה חצים כמו ו' |
|
אם כן נקבצי א'ב' ג'ד'ה' א'ב' ג'ד' ה'ו' מחוברים שוים לשטח ו' בו'[174] והוא כמו מרובע ו' |
|
| |
|
והוא מה שרצינו לבאר |
|
ל"א שני דמיוני נקבץ הנמשכים בדרך המספר מן האחד עד מספר מונח שוים אל המספר המונח מחובר עם מרובעו |
|
ויהיו המספרים הנמשכים מספרי א'ב' ג'ד' ה' |
|
והיה א' אחד |
|
ואומר ששני דמיוני נקבץ מספרי א'ב' ג'ד' ה' שוה למספר ה' ולמרובע ה' |
|
המופת שכאשר חובר נקבץ א'ב'ג'ד'ה' עם נקבץ א'ב'ג'ד' היה העולה שוה למרובע ה' |
|
| |
|
יהיה אם כן נקבץ א'ב'ג'ד'ה' מחובר עם נקבץ א'ב'ג'ד'ה' מוסיף על מרובע[175] ה' כמו מספר ה' המוסף |
|
| |
|
והוא מה שרצינו |
|
ומזאת התמונה יתבאר שנקבץ הנמשכים מן האחד עד מספר מונח שוה לחצי מרובע המספר המונח ולחציו |
|
ל"ב כאשר חוברו נקבצי המספרים הנמשכים מן האחד[176] נמשכים בתכליתם ומתחילים מן האחד עד מספר מונח הנה העולה שוה למרובעי מין המספר המונח הנמשכים בדרך המספר מן האחד[177] עד המספר המונח רצוני שאם היה המספר המונח נפרד[178] יהיה העולה שוה למרובעי הנפרדים הנמשכים עד המספר המונח והאחד עמהם ואם היה המספר המונח זוג יהיה העולה שוה למרובעי הזוגות הנמשכים עד המספר המונח |
|
ויהיה א' [179]אחד |
|
ויחובר עם נקבץ א'ב' ועם נקבץ א'ב'ג' ועם נקבץ א'ב'ג'ד' ועם נקבץ א'ב' ג'ד' ה' ועם נקבץ א'ב' ג'ד' ה'ו' |
|
ויהיה ו' זוג |
|
ואומר שהעולה שוה למרובעי ב'ד'ו' שהם הזוגות |
|
| |
|
המופת שנקבצי[180] א'ב' ג'ד' ה'ו' א'ב' ג'ד' ה' מחוברים שוים למרובע ו' |
|
| |
|
ונקבצי א'ב' ג'ד' א'ב'ג' מחוברים שוים למרובע ד' |
|
ונקבצי א'ב' א'[181] נחברים שוים למרובע ב' |
|
אם כן נחבר נקבצי א' א'ב'[182] א'ב'ג' א'ב'ג'ד' א'ב'ג'ד'ה' א'ב' ג'ד' ה'ו'[183] שוים למרובעי ב'ד'ו' ויהיה האחרון גם כן נפרד |
|
| |
|
ואומר שהעולה שוה למרובעי הנפרדים הנמשכים עד המספר המונח והאחד עמהם |
|
המשל שיהיה האחרון א'ב' ג'ד' ה'ו'ז'[184] ויהיה ז' נפרד |
|
ואומר שהעולה שוה למרובעי א'ג' ה'ז' הנפרדים |
|
| |
|
המופת שנקבצי א'ב' ג'ד' ה'ו' ז' א'ב' ג'ד' ה'ו' מחוברים שוה למרובע ז' |
|
| |
|
ונקבצי א'ב' ג'ד' ה' א'ב' ג'ד' מחוברים שוים למרובע ה' |
|
ונקבצי א'ב'ג' א'ב' מחוברים[185] שוים למרובע ג' |
|
וישאר א' והוא מבואר שהוא[186] שוה למרובעו מפני שהוא אחד |
|
אם כן העולה שוה למרובעי א'ג' ה'ז' |
|
| |
|
והוא מה שרצינו |
|
ל"ג כאשר חוברו נקבצי המספרים הנמשכים מן האחד נמשכים בראשיתם עד שהגיע ההמשך[187] אל האחרון מהם הנה העולה שוה למרובעי כל המספרים ההם |
|
ויחובר נקבץ א'ב' ג'ד' ה' עם נקבץ ב'ג' ד'ה' ועם נקבץ ג'ד'ה' ועם נקבץ ד'ה' ועם מספר ה' |
|
ואומר שהעולה שוה למרובעי א'ב'ג'ד'ה'[188] |
|
| |
|
המופת שכל אחד ממספרי א'ב' ג'ד'ה' הוא באלו המספרים כמספר מה שבו מן האחדים |
|
וזה שכל מספר יהיה מספר המספרים הנמשכים עדיו כמספרו[189] |
|
אבל המספר ימצא בכל אחד מהנקבצים המתחילים מן המספרים[190] הנמשכים עדיו ואיננו בנקבצים המתחילים מהמספרים[191] אשר אחריו |
|
כי הוא בלתי אפשר שימשך המספר הקטן אחר הגדול |
|
הנה אם כן כל מספר[192] ממספרי א'ב' ג'ד' ה' הוא באלו הנקבצים כמנין מה שבו מן האחדים בשוה וזה שוה למרובעו[193] |
|
אם כן אלו הנקבצים מחוברים שוים למרובעי מספרי א'ב' ג'ד'ה' |
|
והוא מה שרצינו |
|
ל"ד כאשר חובר נחבר נקבצי הנמשכים מן האחד נמשכים [194]בראשיתם עד שהגיע ההמשך אל האחרון עם נחבר נקבצי הנמשכים מן האחד נמשכים בתכליתם ומתחילין מן האחד עד שהגיע ההמשך אל המספר הנמשך לפני האחרון אשר זכרנו הנה העולה שוה לשטח המספר האחרון בנקבץ הנמשכים מן האחד עדיו |
|
ויחובר נחבר נקבצי א'ב' ג'ד' ה' ב'ג'ד'ה' ג'ד'ה' ד'ה' ה' עם נחבר נקבצי א' א'ב' א'ב'ג' א'ב'ג'ד' |
|
ואומר שהעולה שוה לשטח ה' בנקבץ מספרי א'ב'ג'ד'ה' |
|
| |
|
המופת שנקבץ הראשון מהנמשכים בראשיתם הוא א'ב' ג'ד'ה' |
|
וא' יחובר עם נקבץ ב'ג' ד'ה' ויהיה א'ב' ג'ד'ה' |
|
וא'ב' יחובר עם ג'ד'ה' ויהיה א'ב' ג'ד'ה' |
|
וא'ב'ג' יחובר עם ד'ה' ויהיה א'ב' ג'ד'ה' |
|
וא'ב' ג'ד' יחובר עם ה' ויהיה א'ב' ג'ד'ה' |
|
הנה אם כן כאשר חוברו הנקבצים הנמשכים בראשיתם עם גילם מהנקבצים הנמשכים באחריתם היה כל אחד מהם שוה לנקבץ א'ב' ג'ד'ה' |
|
אבל מספר נקבצי הנמשכים בראשיתם הוא כמספר אחדי האחרון שהוא ה' |
|
מפני שמספר המספרים הנמשכים מן האחד עד ה' הם כמספר מה שבה מן האחדים |
|
אם כן העולה ימנהו נקבץ א'ב' ג'ד'ה' במספר אחדי ה' |
|
אם כן כבר יוכה נקבץ א'ב'ג'ד'ה'[195] במספר ה' ויהיה שוה אל העולה מזה החבור[196] |
|
והוא מה שרצינו לבאר |
|
ל"ה כאשר חוסרו שני מספרים נמשכים ממרובעיהם הנה הנשאר שוה לשני כפלי מרובע המספר הקטן |
|
ויהיו שני מספרי ד'ה' נמשכים ויהיה ה' הוא הגדול |
|
ואומר כי כשחוסרו ממרובעיהם מספרי ד'ה' היה הנשאר שוה לשני דמיוני מרובע ד' |
|
המופת שמרובע ה' מוסיף על מרובע ד' כפל שטח אחד בד' ומרובע אחד שהוא אחד |
|
אם כן מרובע ה' מוסיף על מרובע ד'[197] שני דמיוני ד'[198] ואחד |
|
אבל שני דמיוני ד' ואחד שוים לד' וה' מקובצים |
|
לפי שה' מוסיף על ד' אחד |
|
אם כן מרובע ה' שוה למרובע ד' ולמספרי ד'ה' |
|
אם כן מרובעי ד'ה' שוים לשני כפלי מרובע ד' ולמספרי ד'ה' |
|
וכאשר גרענו מהם מספרי ד'ה' היה הנשאר שוה לשני דמיוני מרובע ד'[199] |
|
[200]והוא מה שרצינו לבאר |
|
ל"ו כאשר חובר נקבץ[201] הנמשכים מן האחד ונמשכים בראשיתם עד שהגיע ההמשך[202] אל האחרון הנה אם הוסרו ממנו המספרים ההם הנמשכים יהיה הנשאר שוה לכפל מרובעי המין שלפני האחרון הנמשכים עדיו אם זוג זוג ואם נפרד נפרד והאחד עמהם |
|
ויחוברו נקבצי א'ב' ג'ד'ה' ב'ג'ד'ה' ג'ד'ה'[203] ד'ה' ה' ויחוסרו[204] מהעולה מספרי א'ב' ג'ד'ה' מקובצים |
|
| |
|
ואומר שאם היה המספר שלפני האחרון זוג שהנשאר שוה לכפל מרובעי הזוגות הנמשכים עדיו ואם היה המספר שלפני האחרון נפרד הנה הנשאר שוה לכפל מרובעי הנפרדים הנמשכים עדיו והאחד עמהם |
|
ויהיה תחלה זוג כמו הענין במשלינו זה |
|
ואומר שהנשאר שוה לכפל מרובעי הזוגות הנמשכים עד ה' והם ב' ד' |
|
המופת שהעולה שוה למרובעי א'ב' ג'ד'ה' פחות מספרי א'ב' ג'ד'ה' |
|
אבל הנשאר ממרובעי ד'ה' כשחוסר מהם מספרי ד'ה' שוה[205] לכפל מרובע ד' |
|
והנשאר ממרובעי ב'ג' כשחוסר מהם מספרי ב'ג'[206] שוה לכפל מרובע ב' |
|
ומרובע א' הנשאר לוקח כלו בהלקח א' מפני שא' הוא אחד |
|
אם כן הנשאר שוה לכפל מרובעי ד'ב' |
|
ויהיה גם כן המספר שלפני האחרון נפרד |
|
ואומר שהנשאר שוה לכפל מרובעי הנפרדים הנמשכים עד האחרון |
|
ויהיו המספרים א'ב' ג'ד' ה'ו'[207] והמספר הנמשך לו' לפניו הוא[208] נפרד והוא[209] ה' |
|
ואומר שהנשאר שוה לכפל מרובעי הנפרדים הנמשכים עד ו' והם א'ג'ה' |
|
המופת שהעולה שוה למרובעי א'ב' ג'ד' ה'ו' פחות מספרי א'ב' ג'ד' ה'ו'[210] |
|
אבל הנשאר ממרובעי ה'ו' כשלוקח מהם מספרי ה'ו'[211] שוה לכפל מרובע ה' |
|
והנשאר ממרובעי ג'ד' כשלוקח מהם מספרי ג'ד' שוה לכפל מרובע ג' |
|
והנשאר ממרובעי א'ב' כשלוקח מהם מספרי א'ב' שוה לכפל מרובע א' |
|
אם כן הנשאר כלו שוה לכפל מרובעי א'ג'ה' |
|
והוא מה שרצינו |
|
ל"ז כאשר הוכה מספר מונח על נקבץ הנמשכים מן האחד עד המספר הנמשך לו לאחריו הנה העולה שוה למרובעי מין המספר המונח הנמשכים עדיו שלשה פעמים |
|
ויוכה מספר ה' על [212]נקבץ מספרי א'ב' ג'ד' ה'ו' |
|
ואומר שהעולה שוה לשלשה דמיוני מרובעי מין ה' הנמשכים עדיו והם מספרי א' ג' ה' |
|
המופת ששטח[213] ו' בנקבץ א'ב' ג'ד' ה'ו' שוה לנקבצי א' א'ב' א'ב'ג' א'ב'ג'ד' א'ב'ג'ד'ה' מחוברים עם נקבצי א'ב' ג'ד' ה'ו' ב'ג'ד'ה'ו' ג'ד' ה'ו' ד'ה'ו' ה'ו' ו'[214] |
|
| |
|
אבל נקבצי א' א'ב' א'ב'ג' א'ב'ג'ד' א'ב'ג'ד'ה' שוים למרובעי א'ג'ה' |
|
| |
|
ונקבצי א'ב' ג'ד' ה'ו' ב'ג'ד' ה'ו' ג'ד'ה'ו' ד'ה'ו' ה'ו' ו'[215] כשחוסר מהם א'ב' ג'ד' ה'ו' יהיה הנשאר שוה לשני כפלי מרובעי א'ג'ה' |
|
| |
|
אם כן שטח ו' במספרי א'ב' ג'ד' ה'ו' מקובצים שוה לשלשת דמיוני מרובעי א'ג'ה' ולנקבץ א'ב' ג'ד' ה'ו' |
|
| |
|
ולפי ששטח ה' בנקבץ א'ב' ג'ד' ה'ו' ימנהו נקבץ א'ב' ג'ד' ה'ו' במספר אחדי ה' |
|
ושטח ו' בנקבץ א'ב' ג'ד' ה'ו' ימנהו נקבץ א'ב' ג'ד' ה'ו' במספר אחדי ו'ו' מוסיף על ה' אחד |
|
אם כן שטח ו' בנקבץ א'ב' ג'ד' ה'ו' מוסיף על שטח ה' בנקבץ א'ב' ג'ד' ה'ו' כמו נקבץ א'ב' ג'ד' ה'ו' |
|
אבל שטח ו' בנקבץ א'ב' ג'ד' ה'ו' מוסיף על שלשת דמיוני מרובעי א'ג'ה' כמו נקבץ א'ב' ג'ד' ה'ו' |
|
יהיה אם כן שטח ה' בנקבץ א'ב' ג'ד' ה'ו' שוה לשלשת דמיוני מרובעי א'ג'ה' |
|
והוא מה שרצינו לבאר |
|
ומזאת התמונה התבאר שאם הוכה שלישית המספר המונח על נקבץ הנמשכים מן האחד עד המספר הנמשך לו לאחריו שהעולה שוה למרובעי מין המספר המונח הנמשכים עדיו |
|
וזה שכאשר הוכה המספר ההוא המונח בנקבץ ההוא היה העולה שוה לשלשת דמיוני מרובעי מין המספר המונח הנמשכים עדיו |
|
יהיה אם כן שטח שלישית המספר ההוא המונח בנקבץ ההוא שוה לשלישית שלשה כפלי מרובעי מין[216] המספר המונח הנמשכים עדיו שהוא כמו מרובעי המין ההוא הנמשכים עדיו |
|
והוא מה שרצינו לבאר |
|
ל"ח כאשר הוכה מספר מונח פחות שלישית המספר הנמשך לו לפניו על נקבץ הנמשכים מן האחד עד המספר המונח הנה העולה שוה למרובעי כל המספרים הנמשכים מן האחד עד המספר המונח[217] |
|
ויוכה מספר ו' פחות שלישית מספר ה' על נקבץ א'ב' ג'ד' ה'ו' |
|
ואומר שהעולה שוה למרובעי מספרי[218] א'ב' ג'ד' ה'ו' |
|
המופת ששטח ו' במספרי א'ב' ג'ד' ה'ו' מקובצים שוה[219] לנקבצי א'ב' ג'ד' ה'ו' ב'ג' ד'ה' ו'[220] ג'ד' ה'ו' ד'ה'ו' ה'ו' ו' מחוברים עם נקבצי א' א'ב' א'ב'ג' א'ב'ג'ד' א'ב'ג'ד'ה' |
|
| |
|
אבל נקבצי א'ב'ג'ד'ה'ו' ב'ג'ד'ה'ו' ג'ד'ה'ו' ד'ה'ו' ה'ו' ו'[221] שוים למרובעי א'ב' ג'ד' ה'ו' |
|
| |
|
ונקבצי א' א'ב' א'ב'ג'[222] א'ב' [223]ג'ד' א'ב' ג'ד' ה'[224] שוים למרובעי א'ג'ה' |
|
| |
|
אם כן שטח ו' במספרי א'ב' ג'ד' ה'ו' מקובצים שוה למרובעי א'ב' ג'ד' ה'ו' ולמרובעי א'ג'ה' |
|
| |
|
אבל שטח שלישית ה' בנקבץ א'ב' ג'ד' ה'ו' שוה למרובעי א'ג'ה' |
|
וישאר שטח ו' פחות שלישית ה' בנקבץ א'ב' ג'ד' ה'ו' שוה למרובעי א'ב' ג'ד' ה'ו' |
|
והוא מה שרצינו |
|
ל"ט כאשר חוסר מספר מונח ממרובעו[225] הנה חצי הנשאר שוה אל נקבץ הנמשכים מן האחד עד מספר מה הנמשך לפני המספר המונח |
|
וילקח מספר ו' ממרובע ו' |
|
ויהיה המספר הנמשך לו' לפניו מספר ה' |
|
ויהיה חצי הנשאר ממרובע ו' מספר ז' |
|
ואומר שמספר ז' שוה אל נקבץ הנמשכים מן האחד עד מספר ה' |
|
המופת שמרובע ו' שוה לנקבץ א'ב' ג'ד' ה' מחובר עם נקבץ א'ב' ג'ד' ה'ו' |
|
וכאשר חוסר מהמחובר מספר ו' היה הנשאר שוה לנקבץ א'ב' ג'ד' ה' והוא מחובר עם נקבץ א'ב' ג'ד' ה' |
|
אם כן חצי מספר הנשאר שוה לנקבץ א'ב' ג'ד' ה' |
|
והוא מה שרצינו |
|
מ כאשר חובר מספר מה מונח עם חצי הנשאר כשחוסר המספר המונח ממרובעו הנה העולה שוה אל נקבץ הנמשכים מן האחד עד המספר המונח |
|
ויחובר מספר[226] ו' עם חצי הנשאר ממרובעו כשחוסר ממנו מספר ו' |
|
ואומר שהעולה שוה לנקבץ א'ב' ג'ד' ה'ו' |
|
המופת שמרובע ו' שוה לנקבץ א'ב' ג'ד' ה'ו' מחובר עם נקבץ א'ב' ג'ד' ה' |
|
| |
|
וכאשר לוקח מזה ו'[227] וחובר עם חצי הנשאר שהוא נקבץ א'ב' ג'ד' ה' היה[228] העולה א'ב' ג'ד' ה'ו' והם המספרים הנמשכים מן האחד עד ו' |
|
| |
|
והוא מה שרצינו |
|
מ"א המרובע ההווה מנקבץ הנמשכים מן האחד עד מספר מונח הוא שוה למעוקב המספר המונח ולמרובע נקבץ הנמשכים מן האחד עד המספר הנמשך לפני המספר המונח |
|
ויהיה נקבץ הנמשכים נקבץ א'ב' ג'ד' ה' |
|
ואומר שמרובע נקבץ א'ב' ג'ד'ה' שוה למעוקב ה' ולמרובע נקבץ א'ב' ג'ד' |
|
וזה שמעוקב ה' ימנהו ה' כמספר מה שבמרובעו מן האחדים |
|
אבל מרובע ה' שוה לנקבצי א'ב' ג'ד' א'ב' ג'ד' ה' מחוברים |
|
אם כן ה' הוכה בנקבצי א'ב' ג'ד' א'ב' ג'ד' ה' והיה כמו מעוקב ה' |
|
[229]אבל שטח ה' בנקבצי א'ב' ג'ד' א'ב' ג'ד' ה' שוה לשטח ה' בה' שהוא כמו מרובע ה' ולשטח ה' בנקבצי א'ב' ג'ד' א'ב' ג'ד' ה' שהוא כפל שטח ה' בנקבץ א'ב' ג'ד' |
|
| |
|
אם כן מעוקב ה' שוה למרובע ה' ולכפל שטח ה' בנקבץ א'ב'ג'ד'[230] |
|
ואולם מרובע נקבץ א'ב' ג'ד' ה' שוה למרובע ה' ולכפל שטח ה' בנקבץ א'ב' ג'ד' ולמרובע נקבץ א'ב' ג'ד' |
|
| |
|
אם כן מעוקב ה' עם מרובע נקבץ א'ב' ג'ד' שוה למרובע נקבץ[231] א'ב' ג'ד' ה' |
|
והוא מה שרצינו |
|
ואולם האחד אין מספר לפניו אבל מעוקבו[232] שוה אל מרובע הנקבץ עדיו כי היה ההוא בעינו הנקבץ עדיו ומרובע הנקבץ[233] עדיו והוא בעינו מעוקבו וזה מבואר מאד |
|
מ"ב המרובע ההווה מנקבץ הנמשכים מן האחד עד מספר מונח הנה הוא שוה אל המעוקבים ההוים מהנמשכים מן האחד עד המספר המונח |
|
ויהיה הנקבץ[234] נקבץ א'ב' ג'ד' ה' |
|
ואומר שהמרובע ההוה מנקבץ א'ב' ג'ד' ה' שוה למעוקבים ההוים ממספרי א'ב' ג'ד' ה' |
|
המופת שמרובע נקבץ א'ב' ג'ד' ה' שוה למעוקב ה' ולמרובע נקבץ א'ב'ג'ד'[235] |
|
אבל מרובע נקבץ א'ב'ג'ד'[236] שוה למעוקב ד' ולמרובע נקבץ א'ב'ג' |
|
והנה מרובע נקבץ א'ב'ג' שוה למעוקב ג' ולמרובע נקבץ א'ב'[237] |
|
והנה מרובע נקבץ א'ב' שוה למעוקב ב' ולמרובע א' |
|
והנה מרובע א' שוה למעוקב א' |
|
אם כן מרובע נקבץ א'ב' ג'ד' ה' שוה למעוקבים ההווים ממספרי א'ב' ג'ד' ה' |
|
והוא מה שרצינו |
|
מ"ג כאשר היה המספר מונח שוה לנקבץ הנמשכים מן האחד מונחים והיה המספר המונח אמצעי בין הנמשכים מן האחד רצוני שהוא אמצעי בין האחרון מהם ובין האחד הנה מעוקבי הנמשכים המונחים שוים לנפרדי הנמשכים האחרים והאחד עמהם |
|
ויהיה מספר ו' שוה לנקבץ א'ב'ג' |
|
ויהיה ו' אמצעי בין מספרי א'ב' ג'ד' ה'ו' ז'ח' ט'י' כ' הנמשכים מן האחד |
|
ואומר שנפרדי מספרי[238] א'ב' ג'ד' ה'ו' ז'ח' ט'י'כ' שוים למעוקבי א'ב'ג' |
|
המופת שמעוקבי א'ב'ג' שוים למרובע ו' |
|
ונפרדי א'ב' ג'ד' ה'ו' ז'ח' ט'י'כ' שוים גם כן למרובע ו'[239] שהוא האמצעי |
|
אם כן מעוקבי א'ב'ג' שוים לנפרדי א'ב' ג'ד' ה'ו' ז'ח' ט'י'כ' |
|
והוא מה [240]שרצינו לבאר |
|
מ"ד השטח ההווה ממספר מה במספר מה אם חובר אליו מספר אחר מונח מהמספרים ההם הנה העולה ימנהו המספר הנמשך אל המספר הנשאר הנמשך לאחריו כמנין אחדי המספר המונח |
|
ויוכה א' בב' ויחובר עם העולה א' ויהיה ג' |
|
ויהיה המספר הנמשך אחר ב' ד' |
|
ואומר שג' ימנהו ד'[241] במספר אחדי א'[242] |
|
המופת ששטח א' בב' ימנהו א' כשעור אחדי ב' |
|
וכאשר יחובר עמו א' הנה העולה ימנהו א' בשיעור אחדי ב'[243] ותוספת אחד והיא כמספר אחדי ד'[244] |
|
אם כן העולה ימנהו א' כשעור אחדי ד' ולזה ימנהו ד' כשעור אחדי א' |
|
והוא מה שרצינו |
|
מ"ה כאשר היו שלשה מספרים מתחלפים וחובר שטח המספר הגדול ביתרון האמצעי על הקטן עם שטח המספר הקטון ביתרון הגדול על האמצעי הנה העולה ימנהו המספר האמצעי במספר אחדי יתרון הגדול על הקטן |
|
| |
|
ויהיו שלשה מספרי א'ב'ג' מתחלפים |
|
והיה ב' מוסיף על א' בשעור אחדי ד' |
|
ויהיה ג' מוסיף על ב' בשעור אחדי ה' |
|
ואומר ששטח ג' בד' עם שטח א' בה' מקובצים ימנם ב' בשעור אחדי ה'ד' מקובצים שהם יתרון ג' על א' |
|
| |
|
המופת ששטח ג' בד' ימנהו ג' בשעור אחדי ד' |
|
הנה נחלק שטח ד' בב'[245] בדמיוני ג' ויהיו חלקיו השוים לג' מספרי ז'ח' ט'כ' ל'מ'[246] |
|
הנה מספר אלו החלקים הוא כמספר אחדי ד' |
|
וכזה יתחלק שטח ה' בא' בדמיוני א' ויהיו חלקיו השוים לא' מספרי נ'ס' ע'פ'[247] |
|
הנה מספר אלו החלקים הוא[248] כמספר אחדי ה' ולזה יהיה מספר חלקי ז'ח' ט'כ' ל'מ' נ'ס' ע'פ' כמספר אחדי ד'ה' יחד והוא תוספת ג' על א' |
|
הנה נבדיל מז'ח' ז'צ' בשעור ב' וישאר צ'ח'[249] בשעור ה' |
|
וכזה יהיו ט'ו' ל'ת' בשעור ב' |
|
וישאר כל אחד מן ו'כ' ת'מ' בשעור ה' |
|
ונחלוק צ'ח' בדמיון מה שבו מן האחדים ויהיו חלקיו השוים לאחד צ'ק' ק'ח' ומספרם כמספר אחדי ה' |
|
וכזה יתחלק ו'כ' בדמיוני האחד ויהיו חלקיו ו'ר' ר'כ' |
|
ויהיו חלקי ת'מ' השוים לאחד ת'ש' ש'מ' |
|
וכבר היה מספר מספרי ז'ח' ט'כ' ל'מ' כמספר מה שבד' מן האחדים |
|
אם כן מספר אחדי צ'ק' ו'ד' ת'ש' הוא כמספר מה שבד' מן האחדים[250] |
|
הנה יתחברו [251]צ'ק' ו'ר' ש'ת'[252] עם נ'ס' ויהיה שוה לב' |
|
לפי שמספר אחדי צ'ק' ו'ר' ש'ת'[253] הוא כמספר מה שבד' מן האחדים |
|
יהיו א"כ אחדי צ'ק' ו'ר' ש'ת' מקובצים שוים לד'[254] |
|
ונ'ס' שוה לא' |
|
יהיה א"כ נ'ס' מקובץ עם[255] אחדי צ'ק' ו'ר' ש'ת'[256] שוה לא'ד' מקובצים |
|
ואולם א'ד' מקובצים שוים לב'[257] |
|
יהיה א"כ[258] נ'ס' מקובץ עם אחדי צ'ק' ו'ר' ש'ת'[259] שוה לב' |
|
וכזה התבאר שע'פ' מקובץ עם אחדי ק'ח' ר'כ' ש'מ' שוה לב' |
|
וכבר התבאר שמספר מספרי נ'ס' ע'פ' שוה למספר אחדי צ'ק' ק'ח' לפי שכל אחד מהם שוה למספר ה' |
|
אם כן סכום[260] מספרי נ'ס' ע'פ' כמו[261] אחדי צ'ק' ק'ח' והנמשך להם |
|
הנה כבר התבאר ששטח ד' בג' עם שטח ה' בא' ימנם ב' במספר[262] חלקי ז'ח' ט'כ' ל'מ' נ'ס' ע'פ' והוא כמספר ד'ה' מקובצים שהוא תוספת ג' על א' |
|
והוא מה שרצינו לבאר |
|
מ"ו כאשר היו שלשה מספרים מתחלפים והיה הקטון שנים הנה כשחובר עם כפל השטח ההווה ממספר הגדול פחות אחד ביתרון האמצעי על הקטן המספר הגדול ויתרון האמצעי על הקטן ויתרון הגדול על האמצעי הנה זה כלו שוה לכפל שטח ההווה מהאמצעי פחות אחד על הגדול פחות אחד |
|
| |
|
ויהיו המספרים המתחלפים[263] שלשה והם מספרי שנים א' ב' ויהיה ב' הגדול |
|
ויהיה א' מוסיף על שנים מספר ג' |
|
ויהיה א' פחות אחד מספר ז' |
|
ויהיה ב' פחות אחד[264] מספר ד' |
|
ויהיה יתרון ב' על א' מספר ה' |
|
ואומר שכפל[265] שטח ג' בד' מחובר עם מספרי ב' ג' ה' שוה לכפל שטח ז' בד' |
|
המופת ששטח ג' בד' ימנהו ד' כמספר אחדי ג' |
|
ושטח ז' בד' ימנהו ד' כמספר אחדי ז' |
|
וז' מוסיף על ג' אחד |
|
מפני שא' מוסיף על ג' שנים |
|
אם כן יתרון שטח ז' בד' על שטח ג'[266] בד' הוא[267] שטח אחד בד' שהוא ד'[268] |
|
אם כן יתרון שטח ז' בד' על שטח ג' בד'[269] הוא כמספר ד' |
|
ולזה יהיה יתרון כפל שטח ז' בד' על כפל שטח ג' בד' כמו שני כפלי ד' |
|
ואומר שמספרי ב'ג'ד'[270] נחברים שוים לשני כפלי ד' |
|
המופת שמספר ב' הוא מוסיף על מספר ד' אחד |
|
והנה יתרון מספר ב' על מספר א' הוא ה' |
|
אם כן מספרי א'ה' נחברים שוים לב' |
|
ולזה יהיו מספרי [271]ב'א'ה' נחברים שוים לכפל ב' |
|
אבל יתרון כפל מספר ב' על כפל מספר ד' הוא שנים |
|
אם כן מספרי ב'א'ה' שוים לכפל מספר ד' ולשנים |
|
אבל ג' פחות מא' שנים |
|
יהיו אם כן מספרי ב'ג'ה' מקובצים שוים לכפל מספר ד' |
|
וכבר היה יתרון כפל שטח ז' בד' על כפל שטח ג' בד' כמו כפל מספר ד' |
|
אם כן כפל[272] שטח ג' בד' עם מספרי ב'ג'ה' מקובצים שוים לכפל שטח ז' בד' |
|
והוא מה שרצינו לבאר |
|
מ"ז כאשר היו שני מספרים מתחלפים הנה שטח הקטון בגדול עם יתרון הגדול על הקטן שוה לשטח ההווה מהקטן פחות אחד בגדול פחות אחד כשחובר עמו המספר הגדול והמספר הנמשך לו לפניו[273] |
|
ויהיו שני המספרים מספרי א'ב' |
|
ויהיה מספר ג' נמשך לא' לפניו |
|
ומספר ד' נמשך לב' לפניו |
|
ויהיה יתרון ב' על א' מספר ה' |
|
ואומר שמספר ה' מחובר עם שטח א' בב' שוה למספרי ד'ב' מחוברים עם שטח ג' בד' |
|
המופת ששטח ג' בד' כשחובר עמו ד' ימנהו א' כמספר אחדי ד' |
|
לפי שא' מוסיף על ג' אחד |
|
אם כן שטח ג' בד' כשחובר עם ד' שוה לשטח א' בד' |
|
וגם כן הנה מפני שיתרון ב' על א' הוא ה' יהיה ב' שוה לא'ה' נחברים |
|
והנה יהיה שטח א' בד' נחבר עם א' שוה לשטח א' בב' |
|
הנה מפני זה יהיה שטח א' בד' מחובר עם מספרי א'ה' שוה לשטח א' בב' ולמספר ה' |
|
א"כ[274] שטח ג' בד' מחובר[275] עם מספרי ד'ב' שוה לשטח א' בב' ולמספר ה' |
|
והוא מה שרצינו |
|
מ"ח כאשר היו שלשה מספרים מתחלפים והיה הקטון שנים הנה כפל השטח ההוה מהגדול פחות אחד ביתרון האמצעי על הקטן כשחובר עם הגדול[276] ויתרון האמצעי על הקטן[277] ויתרון הגדול על האמצעי הנה העולה שוה לשטח האמצעי פחות אחד בגדול ולשטח הגדול פחות אחד ביתרון האמצעי על הקטן וליתרון הגדול על האמצעי |
|
| |
|
ויהיו המספרים המתחלפים מספרי שנים א' ב' והיה ב' הגדול |
|
והיה א' מוסיף על שנים מספר ג' |
|
וד' הוא הנמשך לא' לפניו |
|
וה' הוא הנמשך לב' לפניו |
|
וז' הוא יתרון [278]ב' על א' |
|
ואומר שכפל שטח ה' בג' מחובר עם מספרי ג' ב' ז' שוה לשטח ד' בב' ולשטח ג' בה' ולז' |
|
המופת שאנחנו נבדיל שטח ג' בה' ומספר ז' המשותפים |
|
ונאמר ששטח ד' בב' שוה לשטח ג' בה' ולמספרי ג'ב' |
|
וזה ששטח ג' בה' מחובר עם ג' שוה לשטח ב' בג' |
|
לפי שמספר ב' מוסיף על ה' אחד |
|
ולזה יהיה שטח ב' בג' מחובר עם ב' שוה לשטח ד' בב' |
|
אם כן שטח ה' בג' מחובר עם מספרי ג'ב' שוה לשטח ד' בב' |
|
אם כן כפל שטח ה' בג' מחובר עם מספרי[279] ג' ב' ז'[280] שוה לשטח ד' בב' ולשטח ג' בה' ולז' |
|
והוא מה שרצינו לבאר |
|
מ"ט כאשר היו שלשה מספרים מתחלפים והיה יתרון הקטן על שנים מספר מונח הנה כפל השטח ההווה מהגדול פחות אחד ביתרון האמצעי על הקטן כשחובר עם שטח הגדול במספר המונח ועם שטח הגדול פחות אחד במספר המונח והתחבר זה כלו עם המספר הגדול ויתרון האמצעי על הקטן ועם יתרון הגדול על האמצעי הנה העולה שוה לכפל שטח ההווה מהאמצעי פחות אחד בגדול פחות אחד |
|
| |
|
ויהיו המספרים המתחלפים[281] מספרי ג' א'ב' ויהיה ג' הוא הקטן וב' הוא[282] הגדול |
|
ויהיה יתרון ג' על שנים מספר ד' |
|
והיה יתרון א' על ג' מספר ה' |
|
והיה מספר ז' נמשך למספר א' לפניו |
|
ומספר ח' נמשך למספר ב' לפניו |
|
והיה יתרון ב' על א' מספר ט' |
|
והיה מספר כ' שוה למספרי ה'ד' מקובצים |
|
והוא מבואר שמספר כ' הוא פחות שנים מא' |
|
לפי שמספרי ג'ה' שוים לא' |
|
וד' הוא פחות מג' שנים |
|
ולזה גם כן יהיה מספר ז' נמשך למספר כ' לאחריו |
|
לפי שמספר ז' הוא פחות מא' אחד לבד |
|
ואומר שכפל שטח ח' בה' כשהתחבר עם שטח ד' בב' ועם שטח ד' בח' והתחבר זה כלו עם מספרי ב'ה'ט' הנה העולה שוה לכפל שטח ז' בח' |
|
| |
|
המופת ששטח ח' בה' כשחובר [283]עמו ה' שוה לשטח ה' בב' |
|
וכאשר חובר שטח ה' בב' עם שטח ד' בב' היה העולה שוה לשטח ההווה ממספרי ה'ד' מקובצים בב' והוא כמו שטח כ' בב' |
|
| |
|
וכשהתחבר עם העולה ב' יהיה העולה שוה לשטח ז' בב' |
|
| |
|
ואולם שטח ח' בה' עם שטח ח' בד' שוה לשטח ה' בד'ה' [284]מקובצים שהוא[285] שטח ח' בב' |
|
| |
|
אם כן כפל שטח ח' בה' עם שטח ד' בב' ועם שטח ח' בד' ועם מספרי ב'ה'ט' שוה לשטח ז' בב' ולשטח כ' בח' ולמספר ט' |
|
| |
|
וא' הוא המספר האמצעי |
|
וכ' הוא יתרונו על הקטן שהוא שנים לפי מה שהונח בתמונה הקודמת[286] |
|
אבל שטח ז' בב' עם שטח כ' בח' ועם מספר ט' שוה לכפל שטח כ' בה'[287] ולמספרי ב'כ'ט' |
|
| |
|
אם כן כפל שטח ח' בה' עם שטח ד' בב' ועם שטח ח' בד' ועם מספרי ב'ה'ט' שוה לכפל שטח כ' בה'[288] ולמספרי ב'כ'ט' |
|
| |
|
אבל כפל שטח כ' בח' עם מספרי ב'כ'ט' שוה לכפל שטח ז' בח' |
|
לפי שמספרי ב'כ'ט' שוים לשני דמיוני ח' |
|
וז' הוא הנמשך לכ' לאחריו |
|
אם כן כאשר חובר עם שטח כ' בח' מספר ח' יהיה שוה לשטח ז' בח' |
|
אם כן כפל שטח כ' בח' עם מספרי ב'כ'ט' שהם שני דמיוני ח' שוים לכפל שטח ז' בח'[289] |
|
| |
|
אם כן כפל שטח ה' בח' עם שטח ד' בב' ועם שטח ח' בד' ועם מספרי ב'ה'ט' שוה לכפל שטח ז' בח' |
|
| |
|
והוא מה שרצינו לבאר |
|
נ כאשר היו שלשה מספרים מתחלפים והיה[290] יתרון הקטן על שנים מספר מונח הנה כפל שטח הגדול פחות אחד ביתרון האמצעי על הקטן עם דמיוני שטחי האמצעי בגדול כמספר מה שבמספר המונח מן האחדים ועם השטח ההווה מיתרון הגדול על האמצעי בקטן פחות אחד ועם המספר הגדול ועם יתרון האמצעי על[291] הקטן הנה כשהתחבר זה כלו יהיה המקובץ ימנהו השטח ההווה מהאמצעי פחות אחד בגדול פחות אחד כמספר מה שבמספר הקטון מן האחדים |
|
ויהיו המספרים המתחלפים מספרי ג' א'ב' והיה ג' הוא הקטון |
|
והיה יתרון ג' על שנים מספר ד' |
|
והיה יתרון א' על ג' מספר ה' |
|
והיה מספר ז' נמשך למספר א' לפניו |
|
ומספר ח' נמשך למספר ב' לפניו |
|
והיה יתרון ב' על א' מספר ט' |
|
והיה מספר כ' נמשך למספר ג' לפניו |
|
ואומר שכפל שטח ח' בה' עם כפלי [292]שטחי א' בב' במנין מה שבד' מן האחדים ועם שטח ט' בב' ועם מספרי ב'ה' ימנהו שטח ז' בח' כמספר אחדי ג' |
|
| |
|
המופת כי בעבור שהיה שטח א' בב' עם ט' שוה לשטח ז' בה'[293] ולמספרי ב'ח' יהיו כפלי ד' משטחי א' בב' עם כפלי ד' ממספרי ט' שוה לכפלי[294] ד' משטח ז' בה'[295] ולכפלי ד' ממספרי ב' וכפלי ד'[296] ממספרי ח'[297] |
|
| |
|
יהיה אם כן כפלי ד' משטחי א' בב' מחובר עם שטח ד' בט' שוה לכפלי ד' משטחי ז' בה'[298] ולשטח ד' בב' ולשטח ד' בח' |
|
| |
|
אבל שטח ט' בכ' מוסיף על שטח ט' בד' מספר ט' |
|
לפי שמספר כ' מוסיף אחד על[299] מספר ד' |
|
אם כן כפלי ד' משטחי א' בב' עם שטח ט' בכ' שוה לכפלי ד' משטחי ז' בח' ולשטח ד' בכ' ולשטח ד' בח' ולמספר ט' |
|
| |
|
וכאשר חברנו שטח ד' בכ' ושטח ד' בח' ומספר ט' עם כפל שטח ח' בה' ומספרי ב'ה' שנשארו בידינו היה בידינו כפל שטח ח' בה' ושטח ד' בכ' ושטח ד' בח' ומספרי ב'ה'ט' אבל כאשר התחבר זה כלו הוא שוה לשני שטחי ז' בח' לפי מה שהתבאר בשלפניה |
|
| |
|
אם כן כפל שטח ח' בה' עם דמיוני שטחי א' בב' במה[300] שבמספר ד' מן האחדים ועם שטח ט' בכ' ועם מספרי ב'ה' ימנהו שטח ז' בח' כמספר אחדי ד' נחבר עם שנים |
|
| |
|
אבל מספר ד' נחבר עם[301] שנים הוא ג' |
|
אם כן כפל שטח ח' בה' עם דמיוני שטחי א' בב' כמו מה שבמספר ד' מן האחדים ועם שטח ט' בכ' ועם מספרי ב'ה' ימנהו שטח ז' בח' כמספר אחדי ג' |
|
| |
|
והוא מה שרצינו |
|
ובכאן התבאר שהעולה מזה המקובץ ימנהו ג' כמספר אחדי שטח ז' בח' |
|
והוא מה שרצינו לבאר |
|
נ"א כאשר היו שלשה מספרים מתחלפים וחובר השטח ההווה מהגדול פחות אחד ביתרון האמצעי על הקטן עם המספר הגדול ועם יתרון האמצעי על הקטן הנה העולה ימנהו המספר הגדול כמספר אחדי המספר הנמשך למספר יתרון האמצעי על הקטן לאחריו |
|
[302]ויהיו השלשה מספרים המתחלפים מספרי א'ב'ג' ויהיה א' הקטן וב' האמצעי וג' הגדול |
|
ויהיה יתרון ב' על מספר א' מספר ד' |
|
ויהיה המספר הנמשך לג' לפניו מספר ה' |
|
והנמשך לד' לאחריו מספר ז' |
|
ואומר ששטח ד' בה' עם מספרי ג' ד' ימנהו ג' במספר אחדי ז' |
|
המופת ששטח ד' בה' כשהתחבר עמו ד' שוה לשטח ד' בג' |
|
וכאשר[303] חובר עם שטח ד' בג' מספר ג' היה העולה שוה לשטח ז' בג' |
|
אם כן שטח ד' בה' עם מספרי ג' ד' שוה לשטח ז' בג' |
|
אם כן שטח ד' בה' עם מספרי ג' ד' ימנהו ג' כמספר אחדי ז' |
|
והוא מה שרצינו לבאר |
|
נ"ב כאשר היו שלשה מספרים מתחלפים הנה אם חובר השטח ההווה מהגדול פחות אחד ביתרון האמצעי על הקטן עם שטח ההווה מהקטון פחות אחד ביתרון הגדול על האמצעי ועם המספר הגדול ועם יתרון האמצעי על הקטן הנה העולה כשהתחבר זה כלו ימנהו האמצעי כמספר אחדי המספר הנמשך אחרי יתרון הגדול על הקטן |
|
ויהיו המספרים המתחלפים מספרי א' ב' ג' ויהיה מספר א' הקטן ומספר ג' הוא הגדול |
|
והיה יתרון ב' על א' מספר ד' |
|
והיה יתרון ג' על ב' מספר ט' |
|
והיה המספר הנמשך למספר א' לפניו מספר ה' |
|
והנמשך למספר ג' לפניו מספר ל' |
|
והיה יתרון ג' על א' מספר ז' |
|
והנמשך למספר ז' לאחריו מספר ח' |
|
ואומר ששטח ד' בל' עם שטח ט'[304] בה' ועם מספרי ג' ד' ימנהו מספר ב' כמספר[305] אחדי ח' |
|
| |
|
המופת ששטח ד' בל' כשחובר עמו ד' הוא שוה לשטח ד' בג' |
|
ושטח ט' בה' כשחובר עמו ג' הוא שוה לשטח ט' בא'[306] ולב' |
|
מפני שמספר ג' שוה למספרי ב' ט' |
|
וכאשר חובר ט' עם[307] שטח ט' בה' היה שוה לשטח ט' בא |
|
א"כ כאשר חובר ב'ט' יחד שהוא ג' עם שטח ט' בה' היה העולה שוה לשטח ט' בא'[308] ולב' |
|
אם כן שטח ד' בל' עם שטח ט' בה' ועם מספרי ג' ד' שוה לשטח ד' בג' ולשטח ט' בא' ולמספר ב' |
|
| |
|
אבל שטח ד' בג' עם שטח ט' בא' שוה לשטח ז' בב' |
|
[309]אם כן שטח ד' בל' עם שטח ט' בה' ועם מספרי ג'ד'[310] שוה לשטח ז' בב' ולמספר ב' |
|
| |
|
אבל שטח ז' בב' כשחובר עמו ב' הוא שוה לשטח ח' בב' |
|
אם כן שטח ד' בל' עם שטח ט' בה' ועם מספרי ג'ד' ימנהו ב' כמספר אחדי ח' |
|
| |
|
והוא מה שרצינו לבאר |
|
נ"ג נרצה שנמצא שלשה מספרים יהיה הראשון עם חלק מונח מהמספרים הנשארים כמו השני עם חלק מונח שני מהנשארים יותר קטן מהחלק המונח הראשון[311] וכמו השלישי עם חלק מונח שלישי מהנשארים יותר קטן מהחלק המונח השני |
|
ויהיו המספרים אשר אלו החלקים נקראים בהם מספרי א' ב' ג' |
|
ויהיה החלק היותר גדול החלק הנקרא בא' והחלק היותר קטן החלק הנקרא בג' |
|
ולזה יהיה המספר היותר קטן מספר א' והמספר היותר גדול מספר ג' |
|
ויהיה יתרון ב' על א' מספר ד' |
|
ויתרון ג' על ב'[312] מספר ז'[313] |
|
והמספר הנמשך לג' לפניו מספר ח' |
|
והמספר הנמשך לב' לפניו מספר ל' |
|
הנה בהכרח שיהיה מספר א' אם[314] שנים אם מוסיף על שנים |
|
ויהיה תחלה שנים |
|
הנה נחבר המספר האחרון והוא ג' עם ד' שהוא יתרון ב' על א' ויהיה העולה בידינו מספרי ג'ד' מקובצים ונשים העולה ה' והוא יהיה המספר הראשון |
|
עוד נחבר ה' עם שני שטחי המספר הגדול פחות אחד ביתרון האמצעי על הקטן והם שני שטחי ד' בח' ונשים העולה ט' והוא יהיה המספר[315] השני |
|
עוד נחבר עם ט' כפל שטח הקטן פחות אחד ביתרון הגדול על האמצעי והוא כפל שטח ז' בא' פחות אחד ונשים העולה כ' והוא יהיה המספר השלישי |
|
|
|
ונאמר שמספרי ה' ט' כ' הם המספרים המבוקשים |
|
המופת שט' שוה לה' ולשני שטחי ד' בח' |
|
וכ' שוה לה' ולשני שטחי ד' בח'[316] ולכפל ז' בא' פחות אחד שהוא שני דמיוני ז' |
|
| |
|
לפי שא' פחות אחד הוא אחד |
|
אם כן חצי מספרי ט'כ' שוה לה' ולשני שטחי ד' בח' ולמספר ז' |
|
אבל ה' שוה למספרי ג'ד' |
|
אם כן חצי מספרי[317] ט'כ' שוה לשני שטחי ד' בח' [318]ולמספרי ג'ד'ז' |
|
אבל שני שטחי ד' בח' עם מספרי ג'ד'ז' שוה לכפל שטח ל' בח' |
|
אם כן חצי מספרי ט'כ' שוה לכפל שטח ל' בח' |
|
אם כן כבר יוכה שנים בשני דמיוני שטח ל' בח' ויהיה העולה שוה למספרי ט'כ' מקובצים |
|
הנה אם כן ט'כ' ימנם א' שהוא שנים כמספר שני דמיוני שטח ל' בח' |
|
ונשים שני דמיוני שטח[319] ל' בח' מספר מ' |
|
הנה מספר מ' הוא חלק הנקרא בא' ממספרי ט'כ' מקובצים |
|
וגם כן הנה ה' כ' שוים לכפל ה' ולשני שטחי ד' בח' ולכפל ז' |
|
אם כן חצי מספרי ה'כ' שוה לה' ולשטח ד' בח' ולז'[320] |
|
ואולם ה' שוה למספרי ג' ד' |
|
אם כן חצי מספרי ה' כ' שוה לשטח ד' בח' ולמספרי ג'ד'ז' |
|
אבל מספרי[321] ג'ד'ז' שוים לשטח א' בח' |
|
לפי שא' הוא שנים |
|
ומספרי ג'ד'ז' שוים לשני דמיוני מספר ח' |
|
אם כן חצי מספרי ה'כ' שוה לשטח ד' בח' ולשטח א' בח' וזה שוה לשטח ב' בח' |
|
לפי שב' שוה לד'א' |
|
אם כן חצי מספרי ה'כ' שוה לשטח ב' בח' |
|
הנה אם כן חצי מספרי ה'כ' ימנהו ב' כמספר אחדי ח'[322] |
|
ולזה ימנה ב' מספרי ה'כ' כמספר אחדי שני דמיוני ח' |
|
ונשים שני דמיוני ח' כמו נ' |
|
הנה מספר נ' הוא חלק הנקרא בב' ממספרי ה'כ' מקובצים |
|
וגם כן הנה יהיו ה' וט' שוים לכפל ה' ולשני שטחי ד' בח' |
|
א"כ חצי מספרי ה'ט' שוה לה' ולשטח ד' בח' |
|
ואולם ה' שוה למספרי ג'ד'[323] |
|
אם כן חצי מספרי ה'ט' שוה לה' ולשטח[324] ד' בח' ולמספרי ג' ד' |
|
ואולם שטח ד' בח' עם מספרי ג'ד' ימנהו ג' כמספר אחדי הנמשך אחר ד' והוא ל' |
|
אם כן חצי מספרי ה'ט' ימנהו ג' כמספר אחדי ל' |
|
אם כן מספרי ה'ט' ימנם ג' כמספר אחדי שני כפלי ל' |
|
ונשים שני כפלי ל' כמו ס' |
|
הנה מספר ס' הוא חלק נקרא בג' ממספרי ה'ט' מקובצים |
|
ונאמר שמספרי ה'מ' מקובצים שוים למספרי ט'נ' מקובצים או למספרי כ'ס' מקובצים |
|
וזה שה'מ' מקובצים שוים[325] לשני דמיוני שטח ל' בח' ולמספר ה' |
|
ומספרי ט'נ' מקובצים שוים לפי מה שהתבאר למספר ה' ולשני דמיוני שטח ד' בח' ולשני דמיוני ח' |
|
הנה יחובר ח' עם שטח ד' בח' ויהיה שוה לשטח ל' בח' |
|
ולזה יהיו שני דמיוני שטח ד' בח' עם שני דמיוני ח' שוים לשני דמיוני [326]ל' בח'[327] |
|
אם כן מספרי ט'נ' מקובצים שוים למספרי ה'מ' מקובצים |
|
וגם כן הנה מספרי כ'ס' שוים לפי מה שקדם למספר ה' ולשני דמיוני שטח ד' בח' ולכפל ל'[328] |
|
הנה מפני שמספרי ז'ב' מקובצים שוים למספר ג' ול' פחות אחד מב' יהיו מספרי ז'ל' מקובצים פחות אחד מג' |
|
אם כן מספרי ז'ל' מקובצים שוים לח' |
|
אם כן מספרי[329] כ'ס' שוים למספר ה' ולשני[330] שטחי ד' בח' ולכפל ז'ל' מקובצים שהוא כמו כפל ח' |
|
| |
|
אבל שני שטחי ד' בח' עם כפל ח' שוים לשני שטחי ל' בח' |
|
אם כן שני[331] מספרי כ'ס' מקובצים שוים גם כן[332] לשני מספרי ה'מ' |
|
הנה כבר מצאנו שלשה מספרים והם מספרי ה'ט'כ' הראשון והוא ה' עם חלק מא' מהנשארים שוה לשני והוא ט' עם חלק מב' מהנשארים ולשלישי והוא כ' עם חלק מג' מהנשארים |
|
והוא מה שרצינו לבאר |
|
ויהיה גם כן מספר א' מוסיף על שנים |
|
ויהיה יתרונו על שנים מספר ד' |
|
ויהיה יתרון ב' על א' מספר ה' |
|
ויתרון ג' על ב' מספר ז' |
|
והמספר הנמשך לא' לפניו הוא מספר ח' |
|
והמספר הנמשך לפני ב' הוא מספר[333] ט' |
|
והמספר הנמשך לפני ג' הוא ל' |
|
ונשאר[334] הדרוש על ענינו |
|
הנה נקח מדמיוני שטח האמצעי בגדול כשעור יתרון הקטן על שנים ונחבר עם העולה המספר הגדול ויתרון האמצעי על הקטן רצוני שנקח מדמיוני שטח ב' בג' כמנין מה שבמספר ד' מן האחדים ונחבר עם העולה[335] מספרי ג' ה' ונשים העולה מספר מ' והוא יהיה המספר הראשון |
|
| |
|
עוד נחבר מספר מ' עם שני שטחי המספר הגדול פחות אחד ביתרון האמצעי על הקטן רצוני שנחבר מ' עם שני שטחי ה' בל' ונשים העולה נ' והוא יהיה המספר[336] השני |
|
עוד נחבר נ' עם שני שטחי הקטן פחות אחד ביתרון הגדול על האמצעי רצוני שנחבר נ' עם שני שטחי ז' בח' ונשים העולה ס' והוא יהיה המספר השלישי |
|
|
|
ואומר שמספרי מ' נ' ס' הם המספרים[337] המבוקשים |
|
המופת שמספר נ' שוה למספר [338]מ' ולשני שטחי ה' בל' |
|
ומספר ס' שוה למספר מ' ולשני שטחי ה' בל' ולשני שטחי ז' בח' |
|
אם כן חצי מספרי נ'ס' שוה למספר מ' ולשני שטחי ה' בל' ולשטח ז' בח' |
|
אבל מספר מ' שוה לכפלי מספר ד' מדמיוני שטח ב' בג' ולמספרי ג'ה' |
|
אם כן חצי מספרי נ'ס' שוה לכפלי ד' מדמיוני שטח ב' בג' ולשני שטחי ה' בל' ולשטח ז' בח' ולמספרי ג'ה' |
|
| |
|
אבל כפלי ד' מדמיוני שטח ב' בג' עם שני שטחי ה' בל' ועם שטח ז' בח' ועם מספרי ג'ה' ימנהו א' כמספר אחדי שטח ט' בל' |
|
| |
|
ולזה ימנה א' מספרי נ'ס' כמספר כפל שטח ט' בל' |
|
ונשים כפל שטח ט' בל' מספר ע' |
|
הנה מספר ע' הוא חלק נקרא בא' ממספרי[339] נ'ס' מקובצים |
|
וגם כן הנה מ'ס' שוים לכפל מ' ולשני שטחי ז' בח' ולשני שטחי ה' בל' |
|
ולזה יהיה חצי מספרי מ'ס' שוה למספר מ' ולשטח ה' בל' ולשטח ז' בח' |
|
אבל מספר מ' שוה לכפלי ד' משטחי ב' בג' ולמספרי ג'ה' |
|
אם כן חצי מספרי מ'ס' שוה לשטח ה' בל' ולשטח ז' בח' ולמספרי ג'ה' ולכפלי ד' מדמיוני שטח ב' בג' |
|
| |
|
אבל שטח ה' בל' עם שטח ז' בח' ועם מספרי ג'ה' ימנהו ב' כמספר הנמשך אחר יתרון ג' על א' והוא המספר הנמשך אחר ה' ז' מקובצים |
|
| |
|
ונשימהו מספר ק' |
|
והנה כפלי ד' מדמיוני שטח ב' בג' ימנהו ב' כמספר אחדי שטח ד' בג' |
|
לפי שכפל ד' מדמיוני שטח ב' בג' הוא מורכב ממספרי ד' ב' ג' |
|
אם כן חצי מספרי מ'ס' ימנהו ב' כמספר שטח ד' בג' וכמספר ק' |
|
ולזה התבאר שמספרי מ'ס' מקובצים ימנם ב' כמספר אחדי כפל שטח ד' בג' וכמספר[340] כפל ק' |
|
ונשים כפל שטח ד' בג' וכפל מספר ק' מספר פ' |
|
הנה מספר פ' הוא חלק נקרא בב' ממספרי מ'ס' מקובצים |
|
וגם כן הנה מ' ונ' שוים לכפל מ' ולשני שטחי ה' בל' |
|
אם כן חצי מספרי מ'נ' שוה לשטח ה' בל' ולמ' |
|
ואולם מספר מ' שוה לכפלי ד' משטח ב' בג' ולמספרי ג'ה' |
|
אם כן חצי מספרי מ'נ' שוה לשטח ה' בל' ולמספרי ג'ה' ולכפלי ד' משטח ב' בג' |
|
אבל שטח ה' בל' עם מספרי ג'ה' ימנהו ג'[341] [342]כמספר אחדי הנמשך אחר ה' |
|
ונשימהו מספר ר' |
|
וכפלי ד' משטח ב' בג' ימנהו ג' כמספר אחדי שטח ד' בב' |
|
אם כן חצי מספרי מ'נ' ימנהו ג' כמספר אחדי[343] שטח ד' בב' וכמספר ר'[344] |
|
אם כן מספרי מ'נ' ימנהו ג'[345] כמספר כפל שטח ד' בב' וככפל מספר ר'[346] |
|
ונשים כפל שטח ד' בב' וכפל מספר ר'[347] מספר צ' |
|
הנה מספר צ' הוא חלק נקרא בג' ממספרי מ'נ' מקובצים |
|
ונאמר שמספרי מ'ע' מקובצים ומספרי נ'פ'[348] מקובצים ומספרי ס'צ'[349] מקובצים שוים קצתם לקצת |
|
המופת שמ'ע' שוים למספר מ' ולכפל שטח ט' בל' |
|
ומספרי נ'פ' שוים למספר מ' ולשני שטחי ה' בל' ולשני שטחי ד' בג' ולכפל מספר ק' |
|
ונשליך מספר מ' המשותף |
|
ונאמר שכפל שטח ט' בל' שוה לשני שטחי ה' בל' מחוברים עם שני שטחי ד' בג' ועם כפל מספר ק' |
|
וזה שיתרון ט' על ה' הוא ח' |
|
לפי שא'ה' מקובצים שוים לב' |
|
ויהיו אם כן ח'ה'[350] מקובצים[351] שוים לט' |
|
אם כן יתרון שטח ט' בל' על שטח ה' בל' הוא שטח ח' בל' |
|
ונאמר שק'ד' שוים לל' |
|
וזה שמספר ק' שוה לה'ז' ולאחד |
|
אבל מספרי ה'ז'א' שוים לג' |
|
אם כן ק'א' מוסיף על ג' אחד |
|
אם כן ק'ח' שוה לג' |
|
ולזה יהיה ק'ח' מוסיף על ל' אחד |
|
אם כן ק'ד' שוה לל' |
|
וכאשר התבאר זה הנה יתבאר שכפל שטח ט' בל' שוה לכפל שטח ה' בל' ולשני שטחי ד' בג' ולכפל מספר ק' |
|
וזה ששטח ד' בל' עם ד' שוה לשטח ד' בג' |
|
א"כ שני שטחי ד' בג' שוים לשני שטחי ד' בל' ולכפל ד'[352] |
|
אם כן שני שטחי ד' בג' וכפל מספר[353] ק' שוים לשני שטחי ד' בל' ולכפל ד'ק' שהוא כמו כפל ל' |
|
| |
|
אבל שני שטחי ד' בל' כאשר חובר עמהם כפל ל' שוים לכפל שטח ח' בל' |
|
אבל כפל שטח ד' בג' עם כפל מספר ק' שוה לכפל שטח ח' בל'[354] |
|
וכאשר חובר עם זה כפל שטח ה' בל' היה העולה[355] שוה לכפל שטח ה' בל' ולכפל שטח ח' בל' |
|
| |
|
אבל כפל שטח ה' בל' עם כפל שטח ח' בל' שוה לכפל שטח ט' בל' |
|
אם כן מספרי מ'ע' מקובצים שוים למספרי נ'פ' מקובצים |
|
וגם כן הנה מספרי ס'צ' שוים למספר מ' ולשני שטחי ה' בל' ועם שני שטחי ז' בח' ולשני שטחי ד' בב' ולכפל מספר ר' |
|
| |
|
ומספרי מ'ע'[356] שוים למספר מ' ולכפל שטח ט' בל' |
|
ונשליך מספר מ' המשותף |
|
ונאמר ששני שטחי ה' בל' ושני שטחי ז' בח' ושני שטחי [357]ד' בב'[358] וכפל מספר ר' שוים לכפל שטח ט' בל' |
|
| |
|
וזה כי לפי שר' מוסיף על ה' אחד |
|
יהיה מוסיף ז'ר' על ז'ה'[359] אחד |
|
אם כן ז'ר' שוה לק' |
|
וכבר התבאר שיתרון שטח ט' בל' על שטח ה' בל' הוא שטח ח' בל' |
|
וכאשר התישב זה כלו הנה נבאר ששני שטחי ז' בח' עם שני שטחי ד' בב' וכפל מספר ר' שוה לכפל שטח ח' בל' |
|
וזה ששטח ז' בד' עם מספר ז' שוה לשטח ח' בז' |
|
יהיו אם כן שני שטחי ז' בח' שוים לשני שטחי ד' בז' ולכפל מספר ז' |
|
וגם כן הנה שטח ד' בב' שוה לשטח ד' בט' ולמספר ד' |
|
אם כן שני שטחי ד' בב' שוים לשני שטחי ד' בט' ולכפל מספר ד' |
|
אם כן שני שטחי ז' בח' עם שני שטחי ד' בב' וכפל מספר ר' שוה לשני שטחי ד' בז'ט' מקובצים ולכפל מספרי ז'ר'ד' |
|
| |
|
ולפי שז'ב' מקובצים[360] שוים לג' יהיו ז'ט' שוים לל' |
|
ולפי שמספרי ז'ר' שוים למספר ק' יהיו מספרי ז'ר'ד' שוים לק'ד' |
|
אבל ק'ד' שוה לל' |
|
אם כן מספרי ז'ר'ד' שוים לל' |
|
אם כן שני שטחי ז' בח' עם שני שטחי ד' בב' וכפל מספר ר'[361] שוה לשני שטחי ד' בל' ולכפל מספר ל' |
|
| |
|
אבל שני שטחי ד' בל' וכפל מספר ל' שוה לשני שטחי ח' בל' |
|
וכאשר חובר זה עם כפל שטח ה' בל' יהיה העולה שוה לשני שטחי ח' בל' ולשני שטחי ה' בל'[362] וזה כבר התבאר שהוא שוה לכפל שטח ט' בל' |
|
| |
|
אם כן מספרי ס'צ' מקובצים שוים למספרי נ'פ'[363] מקובצים |
|
הנה כבר מצאנו שלשה מספרים והראשון והוא מ' עם חלק מא' מהנשארים הוא כמו השני והוא נ' עם חלק מב' מהנשארים[364] וכמו השלישי והוא ס' עם חלק מג' מהנשארים |
|
והוא מה שרצינו לבאר |
|
נ"ד נרצה שנמצא מספר יוסיף חלק מה ממנו או נקבץ חלקים מה ממנו מספר מונח על חלק אחר ממנו או נקבץ חלקים ממנו יותר קטן מן החלק הראשון או מנקבץ החלקים הראשון |
|
ויהיו החלקים אשר מקובצם יותר גדול ב' חלקים מא' במספר הדרוש וג' חלקים מד' בו ואחד מה' בו |
|
והחלקים אשר מקובצם יותר קטן ז' חלקים מח' [365]במספר הדרוש[366] וט' חלקים מכ' בו |
|
ורצינו שנמצא מספר יהיו החלקים הראשונים ממנו מוסיפים על החלקים השניים אשר נקבצם יותר קטן מספר מ' |
|
ויהיה קטן המספר שימנוהו כל אלה המספרים הקוראים לאלו החלקים בכללם והם א'ד'ה' ח'כ' מספר ל' |
|
ויהיו ב' חלקים מא' בו מספר נ' |
|
וג' חלקים מד' מספר ס' |
|
ואחד מה' בו מספר ע' |
|
וכזה יהיה מקובץ החלקים המוסיפים מספרי[367] נ'ס'ע' מקובצים |
|
ויהיו ז' חלקים מח' במספר ל' מספר פ' |
|
וט' חלקים מכ' בו מספר צ' |
|
וכזה יהיה מקובץ החלקים היותר קטן מספרי פ'צ'[368] מקובצים |
|
ויהיה יתרון מספרי נ'ס'ע' מקובצים על מספרי פ'צ' מקובצים מספר ק' |
|
ונשים יחס ל' אל ר' כיחס ק'[369] אל מ' |
|
ונאמר שמספר ר' הוא המספר המבוקש |
|
המופת שאנחנו נשים ב' חלקים מא' במספר ר' מספר ש' |
|
וג' חלקים מד' בו מספר ת' |
|
ואחד מה' בו מספר ו' |
|
וכזה יהיה מקובץ אלו החלקים מספרי ש'ת'ו' |
|
ונשים ז' חלקים מח' במספר ר' מספר י'[370] |
|
וט' חלקים מכ'[371] בו מספר ך' |
|
וכזה יהיה מקובץ אלו החלקים מספרי י'ך' |
|
והוא מבואר שיחס ב' חלקים מא' במספר ל' אל מספר ל' כיחס ב' חלקים מא' במספר ר' אל מספר ר'[372] |
|
לפי שיחס כל[373] אחד מאלו החלקים[374] הוא כיחס ב' חלקים מא' במספר א'[375] אל מספר[376] א' |
|
ולזה יתבאר שיחס ב' חלקים מא' במספר ל' אל ב' חלקים מא' במספר ר' הוא כיחס ל' אל ר' על התמורה |
|
אם כן יחס נ' אל ש' כיחס ל' אל ר' |
|
ובזה התבאר שיחס ס' אל ת' הוא כיחס ל' אל ר' |
|
ושיחס ע' אל ו' הוא כיחס ל' אל ר' |
|
וכאשר קבצנו הנה יחס מספרי נ'ס'ע' מקובצים אל מספרי ש'ת'ו' מקובצים הוא[377] כיחס ל' אל ר' |
|
וכמו כן יתבאר שיחס מספרי פ'ץ' מקובצים[378] אל מספרי י'ך' מקובצים הוא[379] כיחס ל' אל ר' |
|
הנה אם כן יחס מספרי נ'ס'ע' אל מספרי ש'ת'ו' כיחס מספרי פ'ץ' אל מספרי י'ך' |
|
וכאשר המירונו הנה יחס מספרי נ'ס'ע' אל מספרי פ'ץ' כיחס מספרי ש'ת'ו' אל מספרי י'ך' |
|
אבל מספרי נ'ס'ע' מוסיפים על מספרי פ'ץ' |
|
א"כ מספרי ש'ת'ו' מוסיפים על מספרי י'ך'[380] |
|
ונשים יתרון מספרי ש'ת'ו' על מספרי י'ך' מספר ף' |
|
ולפי שהיה יחס נ'ס'ע' אל ש'ת'ו' כיחס ל' אל ר' |
|
ויחס פ'ץ'[381] אל י'ך' הוא גם כן כיחס ל' אל ר' |
|
הנה כאשר הבדלנו יהיה יחס ק' אל ף' כיחס ל' אל ר' |
|
אם כן יחס ק' אל מ' ואל ף' אחד |
|
אם כן מספרי ף'מ' שוים |
|
וכבר היו מספרי ש'ת'ו' [382]מוסיפים על מספרי י'ך' מספר ף' |
|
אם כן מספרי ש'ת'ו' מוסיפים על מספרי י'ך' מספר מ' |
|
הנה כבר מצאנו מספר והוא ר' וב' חלקים מא' בו עם ג' חלקים מד' בו ואחד מה' בו מוסיפים מספר מ' על ז' חלקים מח' בו וט' חלקים מכ' בו |
|
| |
|
והוא מה שרצינו |
|
נ"ה כאשר היה מספר מה מונח עם חלק גדול או נקבץ חלקים גדול ממספר מונח שני פחות מספר מה[383] מהמספר השני עם חלק קטן או נקבץ חלקים קטן מהמספר הראשון הנה כבר אפשר שימצא מספר שלישי יהיה מספר הראשון המונח עם החלק הגדול או נקבץ החלקים הגדול משני המספרים הנשארים שוה למספר השני המונח עם החלק הקטן או נקבץ החלקים הקטן משני המספרים הנשארים |
|
ויהיה המספר הראשון המונח מספר א' |
|
והמספר השני מספר ב' |
|
ויהיה נקבץ החלקים הגדול ג' חלקים מד' ואחד מה' |
|
ונקבץ החלקים הקטן ז' חלקים מח' |
|
ויהיה מספר א' עם ג' חלקים מד' במספר ב' ואחד מה' ממנו פחות מספר ב' עם ז' חלקים מה'[384] במספר א' כמו מספר ט' |
|
ונאמר שכבר אפשר שימצא מספר שלישי יהיה מספר א' עם ג' חלקים מד' ואחד מה' בשני המספרים הנשארים שוה למספר ב' עם ז' חלקים מח' במספרים הנשארים |
|
המופת כי מפני שנקבץ ג' חלקים מד' ואחד מה' יותר גדול מנקבץ ז' חלקים מה'[385] הנה כבר אפשר שימצא מספר יוסיפו ג' חלקים מד' ואחד מה' בו על ז' חלקים מח' בו כמו מספר ט' |
|
ונשים המספר ההוא[386] מספר כ' |
|
ונאמר שמספר כ' הוא המבוקש |
|
המופת שאנחנו נשים ז' חלקים מח' במספר כ' מספר ל' |
|
יהיו אם כן ג' חלקים מד' במספר כ' ואחד מה' בו שוה למספרי ל'ט' |
|
מפני שג' חלקים מד' ואחד מה' במספר כ' מוסיפים ט' על ז' חלקים מח' בו |
|
ונשים מספר א' עם ג' חלקים מד' במספר ב' ואחד מה' במספר ב' שוה למספר מ' |
|
אם כן מספר ב' עם ז' חלקים מח' במספר א' שוה למספרי מ'ט' |
|
וכאשר התישב זה כלו הנה נבאר שמספר א' עם ג' חלקים מד' ואחד מה' במספרי כ'ב' שוה למספר ב' עם ז' חלקים [387]מח' ממספרי א'כ' |
|
וזה שמספר א' עם ג' חלקים מד' ואחד מה' במספר ב' שוה למ'[388] |
|
ואולם ג' חלקים מד' ואחד מה' במספר כ' שוה למספרי ל'ט' |
|
אם כן מספר א' עם ג' חלקים מד' ואחד מה' במספרי כ'ב' שוים[389] למספרי מ'ל'ט' |
|
וגם כן הנה מספר[390] ב' עם ז' חלקים מח' במספר א'[391] שוה למספרי מ'ט' |
|
ואולם ז' חלקים מח' במספר כ' שוה למספר ל' |
|
הנה אם כן מספר ב' עם ז' חלקים מח' במספרי א'כ' שוה למספרי מ'ל'ט' |
|
וכבר היה מספר א' עם ג' חלקים מד' ואחד מה' במספרי כ'ב' שוה גם כן למספרי מ'ל'ט' |
|
אם כן מספר א' עם ג' חלקים מד' ואחד מה' במספרי ב'כ' שוה למספר ב' עם ז' חלקים מח' במספרי א'כ' |
|
והוא מה שרצינו לבאר |
|
נ"ו נרצה שנמצא מספר מה יהיה חלק מה או[392] נקבץ חלקים מה ממנו שוה לחלק מה או לנקבץ חלקים מה מתחלף לחלק הראשון או לנקבץ החלקים הראשון ויהיה לקוח ממספר מה מונח |
|
המשל שאנחנו נרצה שנמצא מספר יהיה חלק מא' בו וב' חלקים מג' בו שוה לד' חלקים מה' במספר ז' המונח |
|
הנה נשים ד' חלקים מה' במספר ז' מספר ח' |
|
ונשים חלק מא' וב' חלקים מג' במספר ז'[393] מספר ט' |
|
ונשים יחס ז' אל כ' כיחס ט' אל ח' |
|
ונאמר שמספר כ' הוא המספר המבוקש |
|
המופת שאנחנו נשים חלק מא' וב' חלקים מג' במספר כ' מספר ל' |
|
והוא מבואר שיחס חלק מא' וב' חלקים מג' במספר ז' אל חלק מא' וב' חלקים מג' במספר כ' הוא כיחס מספר ז' אל מספר[394] כ' |
|
| |
|
אם כן יחס ט' אל ל' הוא כיחס ז'[395] אל כ' |
|
וכבר היה גם כן יחס ט' אל ח' כיחס ז' אל כ' |
|
אם כן יחס ט' אל ח' ואל ל' אחד |
|
אם כן ח' שוה לל' |
|
הנה אם כן חלק מא' וב' חלקים מג' במספר כ' שוה לד' חלקים מה' במספר ז' המונח |
|
והוא מה שרצינו לבאר |
|
נ"ז נרצה שנמצא שני מספרים יהיה האחד עם חלק מה מהשני כמו האחר עם חלק אחר מהמספר הראשון |
|
הנה יהיו המספרים אשר בהם נקראים החלקים האלו מספרי א' ב' |
|
ונרצה שנמצא שני מספרים יהיה[396] האחד עם חלק מא' מהאחר כמו המספר השני עם חלק מב' מהמספר [397]הראשון |
|
הנה נשים מספר הנמשך[398] למספר א' לפניו מספר ג' |
|
והמספר הנמשך למספר ב'[399] לפניו מספר ד' |
|
ונקח שטח ג' בב' ונשימהו ה' והוא יהיה המספר[400] הראשון |
|
ונקח שטח ד' בא' ונשימהו ז' והוא יהיה המספר השני |
|
ונאמר שמספר ה' עם חלק מא' מז' שוה למספר ז' עם חלק מב' ממספר ה' |
|
המופת שחלק מא' מז' הוא ד' |
|
מפני שא' הוכה בד' והיה ז' |
|
וחלק מב' מה' הוא ג' |
|
מפני שב' הוכה בג' והיה ה' |
|
אם כן מספר ה' עם חלק מא' מז' שוה לשטח ג' בב' ולמספר ד' |
|
אבל שטח ג' בב' כשחובר עם ב' ישוה לשטח א' בב' |
|
אם כן שטח א' בב' מוסיף אחד על שטח ג' בב' עם מספר ד' |
|
לפי שב' מוסיף על ד'[401] אחד |
|
אם כן שטח א' בב' מוסיף אחד על מספר ה' עם חלק מא' מז' |
|
וגם כן הנה מספר ז' עם חלק מב' ממספר ה' שוה לשטח ד' בא' ולמספר ג' |
|
אבל שטח ד' בא' כשחובר עם א' שוה לשטח א' בב' |
|
אם כן שטח א' בב' מוסיף[402] אחד על שטח ד' בא' עם מספר ג' |
|
לפי שמספר[403] א' מוסיף על מספר ג' אחד |
|
אם כן שטח א' בב' מוסיף אחד על מספר ז' עם חלק מב' ממספר ה' |
|
ושטח א' בב' גם כן מוסיף אחד על מספר ה' עם חלק מא' במספר ז' |
|
אם כן מספר ה' עם חלק מא' במספר ז' שוה למספר ז' עם חלק מב' במספר ה' |
|
והוא מה שרצינו לבאר |
|
נ"ח נרצה שנמצא שלשה מספרים יהיה המספר הראשון כשחובר עם השלישי ימנהו השני כמספר אחדי מספר מונח ויהיה המספר השני כשחובר עם השלישי ימנהו הראשון כשעור אחדי מספר מונח שני |
|
ויהיו המספרים המונחים מספרי א'ב' |
|
הנה נשים המספר הנמשך למספר א' לאחריו מספר ג' ונשימהו המספר הראשון |
|
ונשים המספר הנמשך למספר ב' לאחריו מספר ד' ונשימהו המספר השני |
|
ונכה א' בב' ונגרע מהעולה אחד ונשים הנשאר ממנו מספר ז' ונשימהו המספר השלישי |
|
ונאמר שמספרי ג' ד' ז' הם המספרים המבוקשים |
|
רצוני שג'ז' מקובצים ימנם ד' כמספר אחדי א' וד'ז' מקובצים ימנם ג' כמספר אחדי ב' |
|
המופת שז' פחות אחד משטח א' בב' |
|
וג' שוה לאחד ולא' |
|
אם כן [404]מספרי ז'ג' שוים לשטח א' בב' ולא' |
|
אבל שטח א' בב' כשחובר עם א' שוה לשטח א' בד' |
|
אם כן מספרי ג'ז' שוים לשטח א' בד' |
|
ולזה ימנם ד' כמספר אחדי א' |
|
וגם כן מפני שז' פחות אחד משטח א' בב' וד' שוה לאחד ולב' יהיו מספרי ד' ז' שוים לשטח א' בב' ולב' |
|
| |
|
אבל שטח א' בב' כשחובר עם ב' הוא שוה לשטח ג' בב' |
|
אם כן מספרי ד'ז' שוים לשטח ג' בב' |
|
אבל שטח ג' בב' ימנהו ג' כמספר אחדי ב'[405] |
|
אם כן מספרי ד'ז' ימנם ג' כמספר אחדי ב' |
|
הנה אם כן מספרי ג'ז' ימנם ד' במספר אחדי א' ומספרי ד'ז' ימנם ג' כמספר אחדי ב' |
|
והוא מה שרצינו לבאר |
|
נ"ט המספר המורכב מהמרובעים ההווים ממספרים מונחים שוה למרובע ההווה מהמספר המורכב מהמספרים המונחים ההם |
|
ויהיו המספרים המונחים א'ב'ג' |
|
ויהיה המספר המורכב ממרובעי מספרי[406] א' ב' ג' מספר ד' |
|
ויהיה המספר[407] המורכב ממספרי א' ב' ג' מספר ה' |
|
ואומר שמספר ד' שוה למרובע ההווה ממספר ה' |
|
המופת כי מפני שכל מספר מרובע מורכב משני דמיוני יסודו הנה[408] יהיה מספר ד' מורכב[409] ממספרי א'א' ב'ב' ג'ג' |
|
ויתבאר לפי מה שקדם ששטח המספר המורכב ממספרי א' ב' ג' במורכב ממספרי[410] א' ב' ג' הוא מספר ד' |
|
אם כן המספר המורכב ממספרי א' ב' ג' והוא ה' הוכה על עצמו והיה ד' |
|
אם כן מספר ד' שוה למרובע ההווה ממספר ה' |
|
והוא מה שרצינו לבאר |
|
ס המספר המורכב ממעוקבי מספרים[411] מונחים שוה למעוקב ההווה ממספר המורכב מהמספרים המונחים ההם |
|
ויהיו המספרים המונחים מספרי א' ב' ג' |
|
ויהיה המורכב ממעוקבי מספרי א'ב'ג' מספר ד' |
|
ויהיה המספר המורכב ממספרי א'ב'ג' מספר ה' |
|
ואומר שמספר ד' שוה למעוקב ההווה ממספר ה' |
|
המופת מפני שכל מעוקב מורכב משלשה דמיוני יסודו יהיה מספר ד' מורכב ממספרי א'א'א' ב'ב'ב' ג'ג'ג' |
|
אם כן מספר ד' שוה לשטח ההווה ממורכב מספרי א'ב'ג' על מורכב מספרי א'ב'ג' א'ב'ג' |
|
אבל מורכב מספרי א'ב'ג' א'ב'ג' שוה לשטח ההווה ממורכב מספרי א'ב'ג' על מורכב מספרי [412]א'ב'ג' שהוא כמו מרובע מספר המורכב ממספרי א'ב'ג' |
|
אם כן מספר ד' שוה לשטח ההווה ממורכב מספרי א'ב'ג' על מרובע מורכב מספרי א'ב'ג' |
|
ולזה יהיה מספר ד' שוה לשטח ההווה ממספר ה' על מרובע מספר ה' |
|
אם כן מעוקב מספר ה' שוה למספר ד' |
|
והוא מה שרצינו לבאר |
|
ס"א כאשר חובר שטח ההווה ממספר מונח במרובע מספר מונח[413] שני עם השטח ההוה מהמונח השני במרובע המספר המונח הראשון הנה העולה שוה לשטח ההווה משטח אחד מהמספרים[414] המונחים באחר על מקובץ המספרים המונחים |
|
ויהיו המספרים המונחים מספרי א'ב' |
|
והיה מרובע מספר א' מספר[415] ג' |
|
ומרובע מספר[416] ב' מספר ד' |
|
וחובר שטח א' בד' עם שטח ב' בג' והיה ה' |
|
והיה שטח א' בב' מספר ז' |
|
ואומר שמספר ה' שוה לשטח ההווה ממספר ז' במספרי א'ב' מקובצים |
|
המופת ששטח א' בד' מורכב ממספרי א' ב' ב' |
|
לפי שמספר ד' הוא מרובע ב' |
|
אם כן שטח א' בד' שוה לשטח ההווה ממורכב מספרי א'ב' ב'ב' |
|
וכבר היה מורכב א'ב' מספר ז' |
|
אם כן שטח א' בד' שוה לשטח ז' בב' |
|
ובזה התבאר ששטח ב' בג' שוה לשטח ההווה ממורכב א'ב' במספר א' |
|
אם כן שטח[417] ב' בג' שוה לשטח ז' בא' |
|
אם כן שטח א' בד' עם שטח ב' בג' שוים לשטח ז' בא' מחובר עם שטח ז' בב' |
|
אבל שטח ז' בא' מחובר עם שטח ז' בב' שוה לשטח ההווה ממספר ז' במספרי א'ב' מקובצים |
|
אם כן שטח א' בד' עם שטח ב' בג' שוה לשטח ז' בא'ב' מקובצים |
|
והוא מה שרצינו לבאר |
|
ס"ב כאשר היו שני מספרים מונחים הנה המעוקב ההווה ממקובצם[418] מוסיף על המעוקב ההווה מהמספר הראשון מהם כמו שלשה דמיוני השטח ההווה משטח המספר הראשון המונח בשני על מקובצם[419] וכמו מעוקב המספר השני |
|
ויהיו המספרים המונחים מספרי א' ב' |
|
והיה שטח א' בב' מספר ג' |
|
והיה מעוקב א' מספר ד' |
|
ומעוקב א'ב' מקובצים מספר ה' |
|
ומעוקב ב' מספר ז' |
|
ואומר שמספר ה' מוסיף על מספר ד' [420]כמו שלשת דמיוני שטח ג' בא'ב' מקובצים וכמו מספר ז' |
|
המופת שא'ב' הוכה על עצמו והיה שוה למרובעי מספרי[421] א'ב' ולכפל שטח א' בב' |
|
אם כן מרובע[422] א'ב' מקובצים שוה למרובעי א'ב' ולכפל מספר ג' |
|
וכבר הוכה מרובעי א'ב' וכפל מספר ג' על מספרי א'ב' מקובצים והיה ה' |
|
לפי שמרובע א'ב' מקובצים יוכה על א'ב' מקובצים ויהיה ה' |
|
ואומר שמספר ה' מוסיף על מספר ד' כמו שלשה דמיוני שטח[423] ג' בא'ב' מקובצים[424] וכמו מספר ז' |
|
וזה שמרובע א' כבר הוכה בא'ב' והיה העולה שוה לשטח א' במרובע א' שהוא מספר ד' ולשטח ההווה ממרובע א' במספר ב' |
|
| |
|
וגם כן הנה מרובע ב' כבר הוכה בא'ב' והיה העולה שוה לשטח ההווה ממרובע ב' בב' שהוא מספר ז' ולשטח ההווה ממרובע ב' במספר[425] א' |
|
| |
|
אם כן מרובעי א'ב' כאשר הוכו על א'ב' היה העולה שוה למספר ד'[426] ולמספר ז' ולשטח מרובע א' במספר ב' ולשטח מרובע ב' במספר א' |
|
| |
|
אבל שטח מרובע א' במספר ב' עם שטח מרובע ב' במספר א' שוה לשטח ג' בא'ב' |
|
אם כן מרובעי א'ב' כאשר הוכו על א'ב' היה העולה שוה למספר ד' ולמספר ז' ולשטח ג' בא'ב' |
|
ואולם מספר ג' כאשר הוכה בא'ב' היה העולה שטח ג' בא'ב' |
|
אם כן כפל ג' כאשר הוכה בא'ב' היה העולה שני דמיוני שטח ג' בא'ב' |
|
אבל כאשר הוכו מרובעי מספרי א'ב' על א'ב' היה העולה שוה למספר ד' ולמספר ז' ולשטח ג' בא'ב' |
|
אם כן כאשר הוכו מרובעי מספרי א'ב' וכפל מספר ג' על א'ב' היה העולה שוה למספר ד' ולמספר ז' ולשלשת דמיוני שטח ג' בא'ב' |
|
| |
|
אבל מרובעי מספרי א'ב' וכפל מספר ג' כאשר הוכו על א'ב' היה העולה ה' |
|
אם כן מספר ה' שוה למספר ד' ולמספר ז' ולשלשת דמיוני שטח ג' בא'ב' |
|
אם כן מספר ה' מוסיף על מספר ד' כמו שלשת דמיוני שטח ג' בא'ב' מקובצים וכמו מספר ז' |
|
והוא מה שרצינו לבאר |
|
וכבר יתבאר מזאת התמונה בעצמה שמעוקב א'ב' שוה למספר ד' ולמספר ז' ולשלשת דמיוני שטח מרובע א' בב' ולשלשת דמיוני שטח מרובע ב' בא'[427] |
|
| |
|
[428]וזה שמעוקב א'ב' שוה למספר ד' ולמספר ז' ולשלשת דמיוני שטח ג' בא'ב' |
|
אבל שטח ג' בא'ב' שוה לשטח מרובע א' במספר ב' עם שטח מרובע ב' במספר א' |
|
ואם כן שלשת דמיוני שטח ג' בא'ב' שוים לשלשת דמיוני שטח מרובע א' בב' ולשלשת דמיוני שטח מרובע ב' בא' |
|
א"כ[429] מעוקב א'ב' שוה למספר ד' ולמספר ז' ולשלשה דמיוני שטח מרובע א' בב' ולשלשה דמיוני שטח מרובע ב' בא' |
|
| |
|
והוא מה שרצינו לבאר |
Combinatorics |
|
Introduction |
הקדמה |
| Two elements differ in the order in which they are combined in two ways: either one precedes the other or the other precedes it. | שני נושאים[430] יתחלפו בסדר בחבורם בשני דרכים אם שיקדים האחד לאחר אם שיקדים האחר לו |
| The diversity of the combinations of elements is in two ways: either they differ in their elements, but are identical in their number, or they differ only in their arrangement. | החלוף בחבורי[431] הנושאים הוא בשני דרכים אם שיתחלפו בנושאיהם וישתתפו בכמות מספרם אם שיתחלפו בסדר לבד |
| When one and the same element is added to two combinations of elements that differ from each other in some way, the [new] combinations are likewise different. | חבור הנושאים המתחלף חלוף מה לחבור נושאים אחד כשחובר עוד עם כל אחד מהם נושא אחד בעינו הנה הם מתחלפים גם כן |
|
והמשל שיהיה חבור א'ב'ג' מתחלף לחבור ב'ג'ד' בנושאים |
|
וחובר עם כל אחד מהחבורים ה'[432] והיו החבורים ה'א'ב'ג' ה'ב'ג'ד' |
|
הנה הם מתחלפים בהם[433] גם כן כמו החלוף הקודם |
|
וכן הענין אם היו החבורים מתחלפים רק[434] בסדר לבד |
|
וזה שאם חובר ה' עם כל אחד מחבורי א'ב'ג' ב'א'ג' ונשאר סדרם כמו שהיה |
|
והיו החבורים ה'א'ב'ג' ה'ב'א'ג' |
|
הנה הם מתחלפים גם כן כמו החלוף הקודם |
| The number of combinations generated from a given number of elements is equal to the number of combinations generated from the same given number of other elements, when the combinations are of the same kind, meaning if the first combinations differ by their elements, the other combinations differ also by their elements, and if the first differ only by the order [of elements], the other differ only by the order [of their elements] as well | מספר החבורים ההווים ממספר מונח מנושאים מה שוה למספר החבורים ההווים מהמספר המונח מנושאים אחרים כשהיו החבורים על דמיון החבורים הקודמים רצוני שאם היו החבורים הקודמים מתחלפים בנושאיהם יהיו החבורים האחרים מתחלפים בנושאיהם ואם היו החבורים[435] הקודמים מתחלפים בסדר לבד יהיו החבורים האחרים מתחלפים בסדר לבד |
Combinatorial Identities |
|
|
ס"ג כאשר חוברו[436] עם חבור נושאים מונח נושאים מתחלפים הנה החבורים מתחלפים בנושאיהם |
|
משל זה שחבור א'ב'ג' נתחבר עם ה' והיה ה'א'ב'ג' ונחבר עם ד' והיה ד'א'ב'ג' |
|
הנה חבורי ד'א' ב'ג' ה'א' ב'ג' מתחלפים בנושאיהם |
|
ס"ד כאשר היו מחברות מספר מונח מנושאים מתחלפים המתחלפות[437] בסדר לבד מספר מה [438]הנה מחברות המספר הנמשך אחר המספר המונח מנושאים מתחלפים המתחלפות בסדר לבד הם כמו שטח מספר המחברות הקודמות[439] במספר הנמשך אחר המספר המונח |
|
ויהיו הנושאים א'ב' ג'ד'ה' ומספרם ז' |
|
והיה המספר הנמשך אחר ז' מספר ח' |
|
והיה מספר מחברות נושאי א'ב'ג'ד'ה' המתחלפות בסדר לבד מספר ט' |
|
ויהיו נושאים א'ב' ג'ד' ה'ו' מוסיפים נושא אחד על מספר נושאי א'ב'ג'ד'ה' ולזה יהיה מספרם מספר ח' |
|
ונאמר שמספר מחברות נושאי א'ב' ג'ד' ה'ו' המתחלפות בסדר לבד הוא כמספר שטח ט' בח' |
|
המופת שכבר יחובר ו' ויושם ראשון עם כל אחת ממחברות א'ב'ג'ד'ה' המתחלפות בסדר ותשארנה המחברות מתחלפות בסדר[440] |
|
ולזה תהיינה המחברות בהיות ו' ראשון מספר ט' |
|
וגם כן הנה מפני שמחברות א'ב'ג'ד'ה' המתחלפות בסדר לבד הם כמספר ט תהיינה מחברות א'ב'ג'ד'ו' ג"כ מספר ט' |
|
וכבר יחובר ה' ויושם ראשון עם כל אחת מאלו המחברות ותשארנה המחברות מתחלפות בסדר לבד ולזה תהיינה[441] המחברות בהיות ה' ראשון כמספר ט' |
|
וכזה התבאר שכל אחד מאלו הנושאים יושם ראשון תהיינה המחברות המתחלפות בסדר לבד בהיותו ראשון כמספר ט' |
|
תהיינה אם כן אלו המחברות בכללם כמו ט' מוכה על מספרם ואולם מספרם הוא ח' |
|
אם כן מספר מחברות א'ב' ג'ד' ה'ו' המתחלפות בסדר לבד הוא כמספר שטח ח' בט' |
|
והוא מבואר שאין בכל אלו[442] המחברות שמנינו שתים דומות |
|
וזה כי בהיות אחד מהנושאים ראשון אין שם[443] שתי מחברות דומות כי המחברות אשר יתחבר עמהם הם מתחלפות וכן תתחלפנה בהתחברו עמהן |
|
ואין ספק שכאשר לא היה הנושא ראשון אחד שהמחברות מתחלפות בסדר |
|
ובהיות הענין כן הוא מבואר שאין באלו המחברות שמנינו שתי מחברות דומות |
|
ונאמר גם כן שאין שם מחברות זולת אלו |
|
שאם היה אפשר הנה תהיה המחברת ההיא מחברת ד'ה'ו'ג'א'ב' |
|
אבל ד' התחבר עם הנשארים בכל מיני חבורם ואחת ממחברות[444] הנשארים ו' ה' א' ג' ב' |
|
אם כן ד'ו'ה'ג'א'ב'[445] היא אחת מהמחברות שמנינו |
|
ואחר שכן הוא רצוני שאין באלו המחברות שתים דומות ואין שם מחברת זולת אלו הנה אם כן מספר מחברות א'ב' ג'ד' ה'ו' המתחלפות בסדר לבד הוא כמו שטח ט' בח' |
|
והוא מה שרצינו לבאר |
| It is proven that the number of permutations of certain elements is equal to the number that is composed of the successive numbers starting from one, whose number is as the number of these elements.
|
[446]ובכאן התבאר שמספר מחברות נושאים מה המתחלפות[447] בסדר לבד הוא כמספר המורכב מהמספרים הנמשכים מתחילים מן האחד מספרם כמספר הנושאים ההם |
|
וזה שמחברות שניים הם שנים וזה שוה למספר המורכב מאחד ושנים |
|
ומחברות השלשה הם כמו השטח ההווה משלשה בשנים וזה שוה למורכב א'ב'ג' |
|
ובזה התבאר לאין תכלית |
|
ס"ה מספר מחברות השנים המתחלפות אם בסדר אם בנושאיהם[448] במספר מונח[449] מנושאים מתחלפים הוא שוה לשטח ההווה מהמספר המונח במספר הנמשך לו לפניו |
|
ויהיו הנושאים א'ב' ג'ד'ה' ויהיה מספרם מספר ז' |
|
והמספר הנמשך לז' לפניו הוא ח' |
|
ונאמר שמחברות השנים המתחלפות אם בסדר אם בנושאיהן מנושאי א'ב' ג'ד'ה' הם כמספר שטח ז' בח' |
|
המופת שכבר יושם א' ראשון ויתחבר עם כל אחד מהנשארים אשר מספרם מספר ח' אם כן המחברות המתחלפות בהיות א' ראשון הם כמספר ח' |
|
ובזה התבאר שכל אחד מאלו הנושאים יושם ראשון ותהיינה המחברות המתחדשות[450] בהיותו ראשון כמספר ח' |
|
אם כן מספר אלו המחברות בכללם כמו מספר[451] ח' מוכה על מספר אלו הנושאים |
|
ואולם מספר אלו הנושאים הוא מספר ז' |
|
תהיינה אם כן אלו המחברות כמספר שטח ז' בח' |
|
ונאמר שאין באלו המחברות אשר מנינו שתי מחברות דומות שלא תתחלפנה אם בסדר ואם בנושאיהן |
|
וזה כי בהיות האחד מהם ראשון אין שם שתי מחברות דומות לפי שהנושאים אשר התחבר עמהם הם מתחלפים |
|
ואין ספק שלא תהיינה דומות אם לא יהיה הנושא הראשון[452] אחד בשניהם כי לכל הפחות יתחלפו בסדר |
|
הנה אם כן אין באלו המחברות שתים דומות |
|
ונאמר שאין שם מחברת זולת אלו אשר מנינו |
|
שאם היה אפשר זה נניח שתהיה המחברת ההיא ג'ה' |
|
ואולם ג' התחבר עם כל אחד מהנשארים ואחד מהנשארים הוא ה' |
|
אם כן אחת מאלו המחברות אשר מנינו היא ג'ה' |
|
אם כן אין שם מחברת זולת אלו אשר [453]מנינו |
|
וכבר התבאר שאין באלו המחברות שתי מחברות דומות |
|
אם כן מספר אלו המחברות הוא כמו מספר שטח ז' בח' |
|
והוא מה שרצינו לבאר |
|
ס"ו כאשר היה מספר מונח מנושאים מתחלפים והיה מספר מחברות מספר מונח שני מאותם הנושאים מתחלף למספר המונח הראשון[454] וקטן ממנו המתחלפות אם בסדר אם בנושאיהן מספר מונח שלישי הנה מספר מחברות המספר הנמשך אחר המונח השני מאלו הנושאים המתחלפות אם בסדר ואם בנושאיהן הם כמספר השטח ההווה מהמספר המונח השלישי ביתרון המספר המונח הראשון על המספר השני |
|
ויהיו הנושאים א'ב' ג'ד' ה'ו'ז' ויהיה מספר אלו הנושאים מספר ח' |
|
ויהיה מספר ט' מתחלף למספר ח' וקטן ממנו |
|
ויהיו[455] מחברות מספר ט' מאלו הנושאים המתחלפות[456] אם בסדר אם בנושאיהן כמספר ל' |
|
והיה מ' נמשך אחרי ט' |
|
והיה יתרון ח' על ט' מספר נ' |
|
ונאמר שמחברות מספר מ' מאלו הנושאים המתחלפות אם בסדר אם בנושאיהן הם כמספר שטח ל' בנ' |
|
המופת שאנחנו נשים אחת[457] ממחברות מספר ט' מאלו הנושאים מחברת א'ב'ג' |
|
והנה הנושאים הנשארים הם נושאי ד'ה'ו'ז' ומספרם כמספר נ' |
|
הנה כבר יושם כל אחד מנושאי ד'ה'ו'ז' הנשארים ראשון עם מחברת א'ב'ג' ותהיינה המחברות מתחלפות ויהיה מספר נושאי המחברת מספר מ' לפי שכבר הוסף על מספר נושאי המחברת הראשונה נושא אחד |
|
ולפי שהיה מספר ד'ה' ו'ז' כמו מספר נ' תהיינה המחברות המתחדשות ממחברת א'ב'ג' כמספר[458] נ' |
|
ובזה התבאר שמספר המחברות המתחדשות עם כל מחברת ממחברות ט' מאלו הנושאים המתחלפות[459] אם בסדר ואם בנושאיהן הם כמספר נ' |
|
ולזה[460] יהיה מספר אלו המחברות בכללם רצוני מספר מחברות מ'[461] מאלו הנושאים כמו מספר נ' מוכה על מספר מחברות ט' מאלו הנושאים |
|
אבל מספר מחברות ט' מאלו הנושאים [462]הוא ל' |
|
אם כן מחברות מ' מאלו הנושאים הוא כמספר שטח נ' בל' |
|
ונאמר שאין בכל אלו[463] המחברות שמנינו שתי מחברות דומות |
|
וזה שהמחברת האחת כבר חוברו עמה נושאים מתחלפים כפעם בפעם |
|
ולזה יחוייב שתהיינה המחברות ההם מתחלפות |
|
ואין ספק שהמחברות המתחלפות לא תהיינה דומות עם איזה נושא שיחוברו |
|
הנה אם[464] כן אין באלו המחברות שתי מחברות[465] דומות |
|
ונאמר שאין שם מחברת זולת אלו אשר מנינו |
|
שאם היה אפשר זה תהיה המחברת ההיא מחברת ו'ד' ב'ז' |
|
אבל מחברת ד'ב'ז' כבר הושם כל אחד מהנושאים הנשארים ראשון עמה ואחד מהנושאים ההם הוא ו' |
|
אם כן מחברת ו'ד'ב'ז' היא אחת מהמחברות[466] אשר מנינו[467] |
|
ואחר שאין שם שתי מחברות דומות באלו המחברות אשר מנינו ואין שם מחברת זולת אלו הנה אם כן מחברות מ'[468] מאלו הנושאים המתחלפות אם בסדר אם בנושאיהם הוא כמספר שטח נ' בל' |
|
והוא מה שרצינו לבאר |
|
ובכאן התבאר שמחברות מספר מונח ראשון ממספר מונח שני מנושאים מתחלפים המתחלפות אם בסדר ואם בנושאיהן הוא שוה למספר המורכב ממספרים נמשכים מספרם כמו המספר המונח הראשון |
|
ויהיה האחרון מהם המספר המונח השני |
|
ויהיה מספר הנושאים מספר ז' |
|
ויהיו מספרי א'ב' ג'ד' ה'ו'ז' נמשכים מתחילים מן האחד |
|
והוא מבואר שמחברות השנים מהם הם כמספר שטח ו' בז' |
|
ואולם המספרים מספרם שנים והם נמשכים והאחרון מהם הוא ז' |
|
ומחברות השלשה מהם הוא כמו השטח ההווה מה' בשטח ו' בז' |
|
לפי שיתרון ז' על שנים הוא ה' |
|
וזה שוה למורכב ה'ו'ז' |
|
ואלו המספרים מספרם שלשה גם כן והם נמשכים והאחרון מהם הוא ז' |
|
וכזה יתבאר בארבעה שמספר המחברות מהם הוא שוה למורכב ד'ה' ו'ז' |
|
וכזה התבאר זה באיזה מספר שיהיה |
|
ס"ז כאשר היה מספר מונח מנושאים מתחלפים והיו מחברות מספר מונח שני מאלו הנושאים המתחלפות בנושאיהם כמו מספר [469]מונח שלישי והיו מחברות המספר המונח[470] השני מנושאים מתחלפים בסדר לבד[471] כמו מספר מונח רביעי הנה מחברות המספר המונח השני מאלו הנושאים המתחלפים אשר מספרם המספר[472] המונח הראשון המתחלפות אם בסדר ואם בנושאיהן הם כמספר השטח[473] ההווה מהמספר המונח השלישי במספר המונח הרביעי |
|
ויהיו הנושאים ההם[474] נושאי א'ב' ג'ד' ה'ו' והיה מספרם ז' |
|
והיו מחברות מספר ח' מהם[475] המתחלפות בנושאיהן כמו מספר ט' |
|
והיו[476] מחברות הנושאים המתחלפים[477] אשר מספרם ח'[478] המתחלפות בסדר לבד כמו מספר ל' |
|
ואומר שמחברות מספר ח' מנושאי א'ב' ג'ד' ה'ו' המתחלפות אם בסדר אם בנושאיהן הם כמספר שטח ט' בל' |
|
המופת שאנחנו נשים אחת ממחברות ח' מאלו ז'[479] הנושאים המתחלפות בנושאיהן מחברת ב'ג'ד'[480] |
|
ויתחדשו[481] ממנה מחברות מתחלפות בסדר לבד כמו מספר ל' |
|
וכזה התבאר שמספר המחברות המתחלפות בסדר המתחדשות מכל מחברת ממחברות ח' מאלו הנושאים המתחלפות בנושאיהן הוא כמו מספר ל' |
|
אם כן מספר אלו המחברות בכללם הם כמספר מחברות ח' מאלו הנושאים המתחלפות[482] בנושאיהן מוכה על ל' |
|
ואולם מספרם הוא ט' אם כן מספר אלו המחברות בכללם הוא כמספר שטח ט' בל' |
|
ונאמר שאין באלו המחברות שמנינו שתים דומות |
|
לפי שבהיות הנושאים אחרים כבר התחלפו המחברות בסדר |
|
והיה מספרם מספר[483] ל' לפי מה שהנחנו |
|
ואין ספק שבהיות הנושאים מתחלפים לא תהיינה המחברות דומות |
|
אם כן אין באלו המחברות אשר מנינו שתי מחברות דומות |
|
ונאמר שאין שם מחברת זולת אלו אשר מנינו |
|
שאם היה אפשר תהיה המחברת ההיא מחברת ו'ד'ב' |
|
אבל כל[484] נושאי ב'ד'ו' התחברו מכל מיני סדורם ואחד ממיני סדורם הוא מחברת ו'ד'ב' |
|
אם כן מחברת ו'ד'ב' היא אחת מהמחברות אשר מנינו |
|
אם כן אין שם מחברת זולת אלו |
|
ובהיות הענין כן רצוני שאין באלו המחברות אשר מנינו שתי מחברות דומות ואין שם מחברת זולת אלו אם כן מספר מחברות ח' המתחלפות אם בסדר ואם בנושאיהן מנושאי א'ב'ג'ד' [485]ה'ו' הוא כמו מספר שטח ט' בל' |
|
והוא מה שרצינו לבאר |
|
ס"ח כאשר היה מספר מונח מנושאים מתחלפים והיה מספר מחברות מספר מונח שני המתחלפות[486] אם בסדר אם בנושאיהן כמו מספר מונח שלישי והיו מחברות המספר המונח השני מנושאים מתחלפים המתחלפות[487] בסדר לבד מספר מונח רביעי הנה מספר מחברות המספר המונח השני ממספר הנושאים[488] המונח המתחלפות[489] בנושאיהן הוא כמספר אחדי מה שימנה המונח הרביעי המונח השלישי |
|
ויהיו הנושאים המתחלפים נושאי א'ב' ג'ד'ה' ויהיה מספרם מספר ז' |
|
ותהיינה מחברות ח' מאלו הנושאים המתחלפות אם בסדר אם בנושאיהן מספר ט' |
|
ותהיינה מחברות הנושאים אשר מספרם ח' המתחלפות בסדר לבד כמו מספר ל' |
|
ונאמר שמספר ט' ימנהו ל' במספר אחדי מחברות ח' מנושאי א'ב'ג'ד'ה' המתחלפות בנושאיהם[490] |
|
המופת שאנחנו נשים[491] מחברות ח' מאלו הנושאים[492] המתחלפות בנושאיהן כמו מספר[493] מ' |
|
אם כן מחברות ח' מאלו הנושאים המתחלפות אם בסדר ואם בנושאיהן כמספר שטח ל' במ' |
|
ואולם מחברות הנושאים אשר מספרם ח' המתחלפות בסדר לבד הם כמספר ל' |
|
והנה[494] מחברות ח' מאלו הנושאים המתחלפות אם בסדר אם בנושאיהן הם כמספר ט' |
|
אם כן מספר ט' שוה לשטח ל'[495] במ' |
|
ולזה[496] ט' ימנהו מספר ל' כמספר אחדי מ' והוא מספר מחברות ח' מאלו הנושאים המתחלפות בנושאיהן |
|
והוא מה שרצינו לבאר |
|
ס"ט כאשר היה מספר מונח מנושאים מתחלפים והיה מספר מחברות מספר מונח שני מאלו הנושאים המתחלפות בנושאיהם כמו מספר מונח שלישי והיה יתרון המספר המונח הראשון על המספר המונח השני מספר מונח רביעי הנה מחברות המספר המונח הרביעי מאלו הנושאים המתחלפות בנושאיהם הם כמו המספר המונח השלישי |
|
ויהיו הנושאים המתחלפים נושאי א'ב' ג'ד' ה'ו'ז' והיה מספרם מספר ח' |
|
והיו מחברות מספר ט' מאלו הנושאים המתחלפות בנושאיהם[497] מספר ל' |
|
ויהיה יתרון ח' על מספר ט' מספר מ' |
|
ואומר שמחברות מספר מ' מאלו הנושאים המתחלפות בנושאיהן הם גם כן כמו מספר ל' |
|
ונבאר תחלה שכאשר היו שתי מחברות מתחלפות מאלו הנושאים [498]שמחברות שאריתם מאלו הנושאים מתחלפות בנושאיהן גם כן |
|
וזה שאנחנו נשים מחברות א'ב' ג'ד' א'ג' ד'ה' מאלו הנושאים מתחלפות בנושאיהן |
|
ואולם שארית מחברת א'ב'ג'ד' מאלו הנושאים היא מחברת ה'ו'ז' |
|
ושארית מחברת א'ג' ד'ה' היא מחברת ב'ו'ז' |
|
ונאמר שמחברות[499] ב'ו'ז' ה'ו'ז' מתחלפות בנושאיהן |
|
וזה שאם היה אפשר זולת זה יהיה ה' הוא ב' |
|
ואם יהיה הענין כן לא תהיינה מחברות א'ב' ג'ד' א'ג' ד'ה' מתחלפות בנושאיהן וכבר הונחו מתחלפות זה שקר |
|
אם כן כבר יחוייב שתהיינה מחברות ה'ו'ז' ב'ו'ז' מתחלפות בנושאיהן |
|
ובזה התבאר ששאריות כל שתי מחברות מתחלפות מהם הם מתחלפות |
|
וכאשר התישב זה הנה נבאר שמספר מחברות מ' מאלו הנושאים המתחלפות בנושאיהם גם כן כמו מספר ל' |
|
וזה שאנחנו נקח לכל מחברת מספר ט' מאלו הנושאים מחברת שארית הנושאים שהוא כמו מספר מ' רצוני שארית הנושאים |
|
אבל המחברות הראשונות מתחלפות בנושאיהן הנה מחברות[500] שאריותיהן מתחלפות בנושאיהן |
|
ולפי שלקחנו לכל מחברת ט' מאלו הנושאים מחברת שאריתה הנה מספר המחברות[501] המתחדשות מהשאריות הוא כמספר המחברות הראשונות |
|
ואולם מחברות ט' מאלו הנושאים המתחלפות בנושאיהן הם כמו מספר ל' אם כן מחברות השאריות שהם מחברות מ'[502] מאלו הנושאים הם כמו מספר ל' גם כן |
|
והוא מבואר שכל מחברות השאריות מתחלפות |
|
ונאמר שאין שם מחברת זולת אלו אשר מנינו ממספר מ' מאלו הנושאים |
|
שאם היה אפשר תהיה המחברת היא מחברת ג'ה'ז' |
|
ונקח שארית הנושאים והיא א'ב'ד'ו' |
|
אבל א'ב'ד'ו' היא אחת ממחברות ט' מאלו הנושאים וכבר לקחנו לכל מחברת מהם שאריתה ושארית זאת המחברת היא מחברת ג'ה'ז' אם כן מחברת ג'ה'ז' היא אחת מהמחברות הנמנות |
|
וכאשר היה זה כן רצוני שאין שם מחברת זולת אלו אשר מנינו ושכל המחברות אשר[503] מנינו הם מתחלפות בנושאיהם אם כן מחברות מספר מ' מאלו הנושאים המתחלפות בנושאיהם הוא מספר ל' |
|
[504]והוא מה שרצינו לבאר |
|
וכבר יתבאר זה במופת אחר |
|
זה שאנחנו נניח שיהיה מספר הנושאים מספר ח' |
|
ויהיו המספרים הנמשכים עד ח'[505] מספרי א'ב' ג'ד' ה'ו' ז'ח' |
|
ונאמר שמחברות מספר מה מאלו הנושאים המתחלפות בנושאיהם הם כמספר מחברות שארית המספר הזה ממספר אלו הנושאים המתחלפות בנושאיהן ויהיה המספר מספר ג' |
|
והיה יתרון ח' על ג' מספר ה' |
|
ואומר שמחברות מספר ג' מאלו הנושאים המתחלפות בנושאיהם הם כמספר מחברות מספר ה' מאלו הנושאים המתחלפות בנושאיהן |
|
וזה שמחברות[506] מספר ג' מאלו הנושאים הם כמספר מה שימנם מורכב א'ב'ג' מורכב ו'ז'ח' |
|
ומחברות מספר ה' ר"ל המתחלפות בנושא לבד מאלו הנושאים הם כמספר מה שימנה מורכב א'ב'ג'ד'ה' מורכב ד'ה'ו'ז'ח' |
|
ואומר שמורכב א'ב' ג'ד' ה' ימנה מורכב ד'ה'ו'ז'ח' כמספר מה שימנה מורכב א'ב'ג' מורכב ו'ז'ח' |
|
וזה שמורכב א'ב' ג'ד' ה' הוא שוה לשטח ההווה ממורכב ד'ה' במורכב א'ב'ג' |
|
ומורכב ד'ה'ו'ז'ח' הוא שוה לשטח ההווה ממורכב ד'ה' במורכב ו'ז'ח' |
|
הנה ד'ה' הוכו בו שני מורכבי א'ב'ג' ו'ז'ח' והיה מזה מורכבי א'ב' ג'ד'ה' ד'ה' ו'ז'ח' |
|
| |
|
אם כן יחס מורכב א'ב' ג'ד'ה' אל מורכב ד'ה'ו'ז'ח' הוא כמו יחס מורכב א'ב'ג' אל מורכב ו'ז'ח' |
|
| |
|
אם כן מורכב א'ב'ג'ד'ה' ימנה מורכב ד'ה'ו' ז'ח' כשעור מה שימנה מורכב א'ב'ג' מורכב ו'ז'ח' |
|
ולזה יהיה מספר מחברות ג' המתחלפות בנושאיהם מאלו הנושאים שוה למספר מחברות ה' המתחלפות בנושאיהם מאלו הנושאים |
|
ובזה התבאר שמחברות איזה מספר שיהיה ממספר מונח מנושאיהם מתחלפים המתחלפות בנושאיהם שוה למספר מחברות שארית המספר המונח מהנושאים ההם |
|
והוא מה שרצינו לבאר |
| By this the first section is complete, Praise be to God the Blessed. | ובכאן נשלם המאמר הראשון תהלה לי' יתברך[507] |
Section Two |
[508]המאמר השני | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Introduction to the Section |
הצעת המאמר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Know that the wise men have compared God in the world of separate intellects with the one among numbers, even if it is an accident. | דע כי החכמים המשילו השם יתעלה בעולם השכלים הנפרדים לאחד במספר ואם הוא מקרה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Since the foundation of all numbers is one; it is the cause of their existence; it is their creator; it is present in all, yet it is not of their species. | לפי שיסוד כל המספרים הוא אחד הוא עלת מציאותם והוא ממציאם והוא עם כלם ואיננו ממינם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| For, it is not a number itself, only by its division, but then it is no longer one. | כי הוא בעצמו איננו ממספר כי אם בהתחלקו ואז איננו אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If its absence is imagined, then all [the numbers] would disappear, but if their absence is imagined, [the one] would not disappear. | אם ידומה העדרו יעדרו כלם ואם ידומה העדרם לא יעדר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| As a number, it has no upper and lower limit, although it is the lower limit of all numbers and, in a certain sense, an upper limit as well. | ואין לו מצד המספר קצה ראשון ולא קצה אחרון עם היות לכל המספרים קצה ראשון וקצה[509] אחרון בפנים מה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If a lower limit is ascribed to the one as a number, that would only be by its division, but then it is no longer one. | ואם יתואר לאחד קצה מצד המספר לא יהיה זה כי אם בהחלקו ואז איננו אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| We have said "as a number", because insofar as its being a line, a surface, or a body, it has limits, namely the points for a line, the lines for a surface, and the surfaces that surround it for a body. | ואמרנו מצד המספר כי מצד היותו קו או שטח או גשם יש לו קצוות והם בקו הנקדות ובשטח הקוים ובגשם השטחים המקיפים בו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| But, as a number, it does not have this, because the line cannot be divided into points and it is not composed of them; and the surface cannot be divided into lines and it is not composed of them. | אמנם אין זה[510] לו מצד המספר כי הקו לא יתחלק לנקודות ולא יורכב מהם והשטח לא יתחלק לקוים ולא יורכב מהם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Thus, all numbers have one origin, from which they are generated and to which they return. | הנה כל המספרים אב אחד לכלם ממנו יצאו אליו ישובו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Numeration |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The resemblance of the ranks to the rank of units | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Because if the units are added up to ten, then ten becomes a unit, and twenty is like two and thirty like three and forty like four and so on, until reaching to a hundred, then it becomes a unit and two hundred is like two and three hundred is like three and so on, until reaching to a thousand and it becomes a unit, and so it goes on to infinity. | כי כאשר יתוספו האחדים עד עשרה ישוב עשרה להיות אחד ויהיו עשרים כשנים ושלשים כשלשה וארבעים כארבעה וכזה ימשך הענין עד שיגיע אל מאה ויהיה הוא אחד ומאתים כשנים ושלש מאות כשלשה וכן ימשך עד שיגיע לאלף והוא ישוב להיות אחד וככה עד אין קץ | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| So it is clear that all numbers end at nine. | הנה התבאר בזה שכל המספרים יכלו אל תשעה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The names of the ranks | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Thus, the one and the following numbers up to nine are called the first rank. | והנה האחד ומה שימשך אליו מן האחדים עד תשעה יקרא המעלה הראשונה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The ten and the following tens up to ninety are called the second rank. | והעשרה ומה שימשך אליו מן העשיריות עד תשעים יקרא המעלה השנית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| One hundred and the following hundreds up to nine hundred are called the third rank. | והמאה ומה שימשך אליו מהמאות עד תשע מאות[511] יקרא המעלה השלישית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| One thousand and the following thousands up to nine [thousands] are called the fourth rank. | והאלף ומה שימשך אליו מן האלפים עד תשעה יקרא המעלה הרביעית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| In this way the units of the rank proceed in proportion to infinity; meaning the ratio of each one of them to the unit of the preceding rank is ten. | ובזה הדרך ימשכו אחדי המעלות מתיחסים עד אין קץ רצוני שכל אחד מהם יחסו אל אחד מהמעלה שלפניו עשרה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| We said: "to infinity", because the number increase as much as it may, that is, as much as you add to it, you can add more, but there will not be a number that is infinite. | ואמרנו עד אין קץ לפי שהמספר [512]יתוסף אל מה שיתוסף ר"ל שכל מה שתוסיף עליו תוכל להוסיף עוד לא שיהיה שם מספר אין תכלית לו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| For, it is clear that every number has an end and its end is the unit through which it is completed. | כי מן המבואר באיזה מספר שיהיה שיש לו תכלית ותכליתו הוא האחד אשר בו ישלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Finally, we say concerning the number that what is infinite, its impossibility is clear in its essence, for the true meaning of the number and its essence is to indicate the limit of the parts it comprises. | סוף דבר אמרנו במספר מה שאין לו תכלית מבואר בנפשו המנעו כי אמתת דבר המספר ומהותו הוא להודיע תכלית חלקי מה שיקיף בו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Furthermore, every number is necessarily either even or odd. | ועוד כי כל מספר הוא אם זוג ואם נפרד בהכרח וזה תכליתו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Therefore, it cannot be the other way around, meaning that [one] can be divided always, as much as it may, as can be said for a line, because we necessarily arrive to one, and stop there. | על כן לא יהיה הענין בהפך רצוני שיתחלק אל מה שיתחלק תמיד כמו שיאמר זה בקו כי היה מן המחוייב שנגיע לאחד ושם נעמד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Yet, the numerical one can still be divided as much as it may, in the sense of the object. | ואולם קרה לאחד המספרי שיתחלק אל מה שיתחלק[513] מצד הנושא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| As the astrologers do when they want to make the calculation accurate: | כמו שיעשו חכמי התכונה בבאם לדקדק חשבון מה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| They divide the first part into sixty parts that are called primes. | יחלקו החלק האחד לששים ויקראו שברים ראשונים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Each of these fractions is divided into sixty parts called seconds. | וכל אחד מהשברים ההם יחלקו לששים ויקראו שניים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Each of the seconds is divided into sixty parts called thirds. | וכל אחד מהשניים יחלקו לששים ויקראו שלישיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| In this way these proportional fractions proceed to infinity, and their foundation, i.e. their beginning is the first rank, meaning its unit. | ובזה[514] הדרך ימשכו אליו השברים המתיחסים עד לאין קץ ויסודם ר"ל התחלותם היא המעלה הראשונה רצוני לומר האחד ממנה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The required number is [found] in one of two ways: by addition or by subtraction, and whatever else there is, is known by itself. | המספר הדרוש הוא באחד משני דרכים אם במחברת ואם במגרעת ומה שהיה ממנו בזולת אלו הוא נודע בעצמו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואשר במחברת הם בשני דרכים אם שנחבר מספרים דומים אם בלתי דומים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואשר בחבור מספרים בלתי דומים הם בשלשה דרכים אם שיתחלפו בכמותם אם שיתחלפו[515] בנושאיהם וישתתפו בכמותם אם שישתתפו בכמותם ובנושאיהם ולא יתחלפו כי אם בסדר לבד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואשר יתחלפו בכמותם הם בשני דרכים אם שיהיו המספרים או המספר שנוסיף ידועים או בלתי ידועים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואשר הם בלתי ידועים הם בשני דרכים אם שיתוספו בשעור שוה רמוז אליו ויהיה ז' כשיהיו נמשכים או שיתוספו בשעור בלתי רמוז אליו אבל יהיו מתיחסים רצוני שיהיה יחס זה אל זה כיחס זה אל זה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואשר במגרעת בשני דרכים אם שנגרע מספר או מספרים ממספר או שנחלק מספר על מספר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואשר הוא בחלק מספר על מספר הוא בשני דרכים אם שיהיה המספר הנחלק [516]עליו ידוע אם[517] בלתי ידוע כהוצאת שרשי המרובעים והמעוקבים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אלו הם החלקים הפשוטים אשר תפשטם[518] החלוקה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| There is one operation that is used with most of these species, or with all of them, which is to extract the number whose ratio to a number is as the ratio of a given number to a given number, and any similar extraction of the unknown from the known. | וכבר תהיה שם מלאכה ישתמשו בה ברוב אלו המינים או בכלם והיא הוצאת המספר אשר ערכו למספר מה כערך מספר מונח אל מספר מונח ומה שידמה לזה מהוצאת הנעלם מן הידוע בזה האופן | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| We shall explain these operations and the ways, by which the sought is obtained, in this section with God's help. | ואנחנו בג"ה נבאר ענין אלו המלאכות והדרכים אשר בהם יושג הדרוש בהם בזה המאמר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| We have divided this section into six chapters in accordance with this investigation: | וחלקנו זה המאמר לפי זאת החקירה לששה שערים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
השער הראשון בהוסיף מספר או מספרים ידועים למספר ובגרוע מספר[519] או מספרים ידועים ממספר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
השער השני בחבור מספרים דומים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
השער השלישי בחבור מספרים נמשכים או מתיחסים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
השער הרביעי בחבור מספר מנושאים מה תתחלפנה המחברות בנושאיהם או בסדרם לבד או בשניהם יחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
השער החמישי בחלק מספר על מספר היה שיהיה המספר שיתחלק עליו ידוע או בלתי ידוע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
השער הששי בערכין | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Chapter One on the Addition of Numbers to one another and the Subtraction of Numbers from one another |
השער הראשון בחבור המספרים קצתם עם קצת ובמגרעת המספרים קצתם מקצת | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Addition |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| It has already been clarified that all numbers end at 9. | כבר התבאר כי כל המספרים יכלו אל תשעה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Therefore, the number that is at the end of any rank is nine. | ואחר שכן הוא הנה יהיה המספר אשר בתכלית המעלה אחת מן המעלות הוא תשעה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The addition of numbers that do not exceed nine is of the first knowledge for anyone who has an intellect. | וחבור המספרים שלא יעברו תשעה קצת עם קצת הוא מן הידיעות הראשונות לכל בעל שכל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| When you wish to add up numbers, as many as they may be, you should write each number of them in a row and divide the rows into rubrics. | כאשר תרצה לחבר מספרים כמה שיהיו ראוי שתכתוב כל מספר ומספר מהם בטור אחד ותחלק הטורים לאבנים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| In the first rubric you write what is in the first rank of that number. | האבן הראשה תכתוב בה מה שבמספר ההוא מהמעלה הראשונה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If there is nothing in its the first rank, you write a zero there, to indicate that there is no number in this rank. | ואם אין בו מהמעלה הראשונה מאומה תכתוב בה גלגל להורות שאין בזאת המעלה שום מספר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| In the second rubric you write what is in the second rank of that number. | והאבן השנית תכתוב בה מה שבמספר ההוא מהמעלה השנית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If there is nothing in this rank, you write a zero there. | ואם אין בו בזאת המעלה מאומה תעשה שם גלגל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| In the third rubric you write what is in the third rank of that number. | והאבן השלישית תכתוב בה מה שבמספר ההוא מהמעלה השלישית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| And so on endlessly. | ובזה הדרך עד אין קץ | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| You write the rows one beneath the other. | ותעשה הטורים איש [520]תחת אחיו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| And the rubrics are one corresponding the other in all the rows. | והאבנים תהיינה אשה נגדה מכוונות לכל הטורים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| When this is done, you write the result of the addition of these numbers beneath these rows in a row in the appropriate places corresponding to the ranks. | וכאשר ישלם לך זה כתוב[521] העולה בחברך אלו המספרים תחת הטורים ההם בטור אחד במקומות הראויות לו לפי מעלותיו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The way you should go about when adding these numbers up: | הדרך תלך בה בחבור אלו המספרים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Add up what is in all the rows in the first rubric. | חבר מה שבכל הטורים באבן הראשה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If the sum is greater than ten, convert the tens into units of the second [rank], because every unit of it is ten units of the first rank. | ואם עלה יותר[522] מעשרה תעשה מהעשיריות אחדים בשנית כי היה כל אחד ממנה עשרה מאחדי המעלה הראשונה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Write the remainder in the row that is beneath all the rows in the first rubric; it is what we call the row of the result. | והנשאר תכתוב בטור אשר תחת כל הטורים באבן הראשה גם כן והוא אשר נקראהו טור העולה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Then, add up what is in the second rubric of all rows. | אחרי כן תשוב לחבר מה שבאבן השנית בכל הטורים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If the sum is greater than ten, convert the tens into units of the third [rank]. | ואם עולה יותר מעשרה תעשה מהעשיריות אחדים בשלישית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Write the remainder in the second rubric of the row of the result. | והנשאר תכתוב באבן השנית בטור העולה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Then, add up what is in the third rubric of all the rows. | אחר כן תשוב לחבר מה שבאבן השלישית בכל הטורים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Write the sum in the row of the result in the manner we have mentioned. | ותכתוב העולה בדרך שזכרנו בטור העולה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| And so on until all the numbers in all the ranks have come to an end. | וככה עד כלות כל המספרים שבכל המעלות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| When this has been completed, it is clear that the result is the sum of all the numbers, because all the parts of the one are already added to all the parts of the other and the whole is equal to [the sum of] all its parts. | וכאשר ישלם זה הוא מבואר שהעולה הוא המקובץ מכל המספרים כי כבר חוברו חלקי זה בכללם עם חלקי זה בכללם וכלל הדבר שוה לכל חלקיו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון נרצה לחבר מאתים ותשע עם שלשת אלפים ושמנים ותשע ועם ז' אלפים ושש מאות ושלשים ותשע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונכתבם בג' טורים בזאת הצורה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הטור הראשון ט' בראשון גלגל בשנית ב' בשלישית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הטור השנית ט' בראשונה ח' בשנית גלגל בשלישית ג' ברביעית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הטור השלישי ט' בראשונה ג' בשנית ו' בשלישית ז' ברביעית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חברנו מה שבראשונה בכל הטורים ועלה כ"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונכתב בטור העולה[523] ז' בראשונה והכ' תהיינה ב' בשנית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חברנו מה שבשנית מכל הטורים ועלה י"א וב' שנשארו לנו שם והנה י"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונכתוב בטור העולה ג' בשנית והעשרה תהיינה א' בשלישית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חברנו מה שבאבן השלישית בכל הטורים ועלה ח' וא' שנשאר לנו שם והנה ט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונכתבם בטור העולה באבן השלישית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חברנו מה שבמעלה הרביעית בכל הטורים ועלה עשרה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
על כן נכתוב גלגל בטור [524]העולה מרביעית ומהעשרה נכתוב אחד בחמישית בטור העולה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ופה נשלם חבור חלקי אלו המספרים קצתם עם קצת והנה העולה בחברך אלו המספרים הוא רבוא ותשע מאות ול"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Apply this. | והקש על זה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If you want to add up fractions to fractions, and the fractions are sexagesimal fractions: | ואם תרצה לחבר שברים עם שברים ויהיו השברים משברי חכמי התכונה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Write the fractions of one number in a row according to their ranks. | כתב השברים ממספר האחד בטור אחד כפי מדרגתם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| I mean that if they are primes, write them in the last rubric of the row, opposite to what you did with integers. | רצוני שאם הם ראשונים תכתבם באבן האחרונה שבטור הפך מה שעשית בשלמים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If they are not primes, write a zero there. | ואם אינם ראשונים תכתב שם גלגל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Write the seconds in the second [rubric] backwards. | והשניים תכתוב בשנית לאחרונה לאחור | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The thirds in the third [rubric] backwards. | והשלישיים[525] בשלישית לאחור לאחרונה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| And so on, until you have finished writing down all the fractions that are in the first number. | וכן עד השלימך לכתוב כל השברים שבמספר האחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Do likewise with the fractions of all the numbers - write them, each one in its place, in this way. | וכן תעשה לשברי כל המספרים תכתבם איש על מקומו בזה הדרך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| This is so, because the rule of the ranks of fractions is opposite to that of the ranks of integers. | והיה זה כן כי מנהג מדרגות השברים הם בהפך מדרגות השלמים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| For in the ranks of integers there is only one end, which is the lowest rank, but in the case of the fractions it is the other way round, as the end that is found for them is the highest [rank], hence we should begin from it. | כי מדרגת השלמים[526] ימצא בהם הקצה האחד לבד והוא המדרגה היותר מעטה ובשברים ימצא הענין בהפך כי הקצה הנמצא בהם הוא היותר גדולה ולזה ראוי שנתחיל ממנה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Since, according to the previous order the highest rank was after the lowest [rank] and the primes were of the highest rank, so it is appropriate that the primes will be in the last rank of the fractions. | ולפי שעל הסדר הקודם היתה המדרגה היותר גדולה אחר היותר[527] מעטה והיו הראשונים מהמדרגה היותר גדולה במוחלט ראוי שיהיו ראשונים במדרגה האחרונה מהשברים ההם במוחלט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| When you have finished that, add up everything that you find in all the rows in the rank the lowest fractions. | וכאשר ישלם לך זה חבר כל מה שתמצא בכל הטורים במדרגות השברים היותר דקים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If the result is greater than sixty, subtract [the sixty] from it; convert the sixty into one in the rank that is second to it, and write the remainder in that rank in the row of the result. | ואם עלה יותר מששים גרעם מהם ומהששים תעשה אחד במדרגה השנית לה והנשאר כתב במדרגה ההיא בטור העולה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| And so on, until you have added up all the fractions. | וככה עד חברך כל השברים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| When you add up the primes, if the result is in greater than sixty or sixty, convert the sixty into one integer in the row of the result. | ובחברך הראשונים אם עלה יותר מששים או ששים תעשה מהששים אחד שלם בטור העולה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון נרצה לחבר נ"ו שנים ול' שלישים עם כ' ראשונים ומ' שניים ול' שלישיים ועם מ"ו ראשונים כ"ז שניים כ"ה שלישיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכתבנום בג' טורים בזאת הצורה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הטור הראשון גלגל באחרונה נ"ו בשנית ל' בשלישית לאחור | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הטור השני כ' באחרונה מ' בשנית לה ל' בשלישית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הטור השלישי מ"ו באחרונה כ"ז בשנית לה כ"ה בשלישית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
[528]והנה המדרגה אשר שבריה יותר דקים באלו הטורים היא השלישית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חברנו מה שבכל אלו הטורים בשלישית[529] ועלה פ"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נגרע מהם ס' ונשארו כ"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותכתבם[530] בטור העולה תחת המדרגה השלישית והס' שגרענו יהיה[531] א' במדרגה השנית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד חברנו מה שבכל הטורים אשר בשנית עם הא' שנשאר לנו שם ועלה קכ"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונכתב ד' בטור העולה והק"ב תהיינה ב' באחרונה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חברנו מה שבכל אלו הטורים באחרונה עם הב' שנשארו לנו שם והנה ס"ח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונכתוב ח' בטור העולה באחרונה והס' יהיה אחד שלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונכתבהו אחר האחרונה ונעשה שם רושם אחד יבדיל בין השלמים לשברים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה העולה הוא אחד שלם ח' ראשונים ד' שניים כ"ה שלישיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Apply this. | והקש על זה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If there are integers and fractions among the numbers you want to add up: | ואם יהיה במספרים שבאת לחברם שלמים ושברים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Write first the integers in the way I have shown you, and draw a mark between the rank of the units and fractions, so as not to be confused. | תכתוב תחלה השלמים בדרך שהראיתיך ותעשה רושם בין מעלת האחדים לשברים כדי שלא יתבלבל עליך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Then write first the units of the primes and before the primes the seconds, and so on as above. | ותכתוב קודם האחדים השברים הראשונים ולפני הראשונים השניים וכן מה שהגיעו השברים כמו שקדם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| When you have finished that, start adding up from the smallest fractions, adding fractions to fractions and integers to integers, as we have mentioned, and write the result in a row beneath these rows in the corresponding places. | וכאשר ישלם לך זה תחל לחבר מהשברים היותר דקים ותחבר השברים עם השברים והשלמים עם השלמים בדרך שזכרנו ותכתוב העולה בטור אחד תחת אלו הטורים במקומותם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| I shall give you an example how you should write the row in which there are fractions and integers: | ואתן לך משל איך תכתוב הטור שבו שברים ושלמים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם רצית לכתוב מאתים ושלשים שלמים ל"ז שניים מ"ד רביעים מ"ה חמישיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה השלמים יהיו גלגל בראשונה ג' בשנית ב' בשלישית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותעשה רושם בין הראשונה והשברים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותכתוב אחר הרושם קודם הראשונה מהשלמים גלגל לפי שאין בזה המספר ראשונים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ובשנית לה לאחור תכתוב ל"ז שהם שנים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ובשלישית לה לאחור תעשה גלגל לפי שאין בזה המספר שלישיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וברביעית לה לאחור תכתוב מ"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ובחמישית לה לאחור תכתוב מ"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וזאת היא הצורה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| You already know the addition itself from the above. | ואולם החבור הנה כבר ידעתו ממה שקדם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Subtraction |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If you wish to subtract a number from a number, write the number you [want to] subtract from in a row according to its ranks, and beneath it in another row the number you want to subtract. | אם רצית לגרוע מספר ממספר כתב המספר שממנו תגרע בטור אחד כפי מדרגותיו ותחתיו תכתוב בטור אחר המספר שרצית [532]לגרוע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Then, see which rank is the lowest in all the rows, and start subtracting from the lowest rank of the top row what corresponds it in the lower row; write the remainder in the row of the result, in the same rank. | והנה תראה איזו מדרגה היא יותר דקה בכל הטורים ותחל לגרוע במה שבמדרגה היותר דקה בטור העליון מה שכנגדה בטור התחתון והנשאר תכתוב בטור העולה במדרגה ההיא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If there is not enough there to subtract and you are dealing with fractions, lower one unit from the next rank, so they are sixty in that [rank]; then you can subtract whatever you want. | ואם לא היה שם די לגרוע אם היית בשברים הורד אחד במדרגה הבאה אחריה אליה יהיו ששים בה ואחר תוכל לגרוע מה שתרצה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If you are dealing with integers, lower one from the next rank, so they are ten in that [rank]; then you can subtract whatever you want. | ואם היית בשלמים הורד אחד מהמדרגה הבאה אחריה אליה יהיו עשרה בה ואחר תוכל לגרוע מה שתרצה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| You do so until the whole bottom row is subtracted from the top row. | וכן תעשה עד שיגרע כל הטור התחתון מהטור העליון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Write the remainder in its [appropriate] places, in the row of the result. | והנשאר תכתוב כפעם בפעם בטור העולה במקומותיו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| When it is complete, it is clear that you have already subtracted the whole bottom number from the top number. | וכאשר ישלם זה הוא מבואר שכבר גרעת המספר התחתון בכללו ממספר העליון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| For the parts of a thing in their totality are equal to the whole thing. | כי חלקי הדבר בכללם שוים לכל הדבר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| It is necessary that the row, from which you subtract, is a greater number than the number in the other row, for it is impossible to subtract the larger from the smaller. | וראוי שיהיה הטור שתגרע ממנו רב הכמות מהטור השני כי אי אפשר לגרוע הרב מהמעט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון נרצה לגרוע מאתים ושש ונ' ראשונים ל"ז שלישים מל"א אלפים ושמנים ומ"ו שניים ל"ה שלישיים מ"ז רביעים כ"ג ששיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| We write the number from which we subtract in the upper row and the number we want to subtract in the bottom row by their ranks and we make a mark between the fractions and the integers. | והנה כתבנו המספר שממנו נגרע בטור הטור העליון והמספר שרצינו לגרוע בטור התחתון במקומותיהם ועשינו רושם בין השברים לשלמים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The lowest rank are sixths; it is in the upper row 53 sixths. | והנה המדרגה היותר דקה שהיא ששים והיא שכנגדה בטור העליון היא כ"ג ששים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| We subtract from them what corresponds to them in the bottom row, but there is nothing there, so we write 53 in the row of the result in the rank of the sixths.
|
ונגרע מהם מה שכנגדם בטור התחתון ואין שם בטור התחתון דבר על כן נכתב כ"ג בטור העולה במדרגת הששיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Then, we subtract from the zero that comes after 53 what corresponds to it in the bottom row, but there is nothing there in the bottom row, so we write a zero in the row of the result in the rank of the fifths.
|
נשוב לגרוע מהגלגל הבא אחר כ"ג מה שכנגדו בטור התחתון ואין שם בטור התחתון[533] דבר על כן נכתב גלגל בטור העולה במדרגת החמישיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| We subtract from the 47 what corresponds to it in the bottom row, but there is nothing there, so we write in the row of the result 47 in the rank of the fourths.
|
נשוב לגרוע ממ"ז מה שכנגדו בטור התחתון ואין שם בו דבר ולזה נכתב מ"ז בטור התחתון במדרגת הרביעים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| We subtract from the 35 in the top row what corresponds to it in the bottom row; 37 corresponds to it, but we cannot subtract 37 from 35, so we take one from the rank that comes after 35; it becomes 60 in that rank. We add them to 35; it is 95. We subtract 37 from it; 58 remain. We write them in the row of the result in the rank of the thirds.
|
נשוב לגרוע מל"ה שבטור העליון[534] מה שכנגדו בטור התחתון והנה כנגדו ל"ז ולא נוכל לגרוע מל"ה ל"ז על כן נקח אחד מהמדרגה [535]הבאה אחר ל"ה ויהיו ששים בה נחברם עם ל"ה ויהיה צ"ה נגרע מהם ל"ז נשארו נ"ח וכתבנום בטור העולה במדרגת השלישיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Therefore only 45 remain in the next rank. We subtract from them what corresponds to them in the bottom row, but there is nothing there, so write 45 in the row of the result in the rank of the seconds.
|
ולזה נשארו במדרגה הבאה אחר ל"ה מ"ה נגרע מהם מה שכנגדם בטור התחתון והנה אין שם בו דבר ולזה נכתב מ"ה בטור העולה במדרגת השניים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| We turn to subtract from the zero what corresponds to it in the bottom row, but there is 50 corresponding it, and we cannot subtract 50 from a zero. There is no number also in the rank next to it of the integers, to lower to it. But in the rank that is third to it there is the number 8. We lower one from it to the preceding rank. We write 7 above the 8, and the one that we lowered becomes ten in the first rank.
|
נשוב לגרוע מגלגל מה שכנגדו בטור התחתון והנה כנגדו נ' ולא נוכל לגרוע מהגלגל נ' וגם במדרגה הסמוכה לה מהשלמים אין מספר מה להוריד אליה ואולם בשלישית לה הוא מספר ח' נוריד מהם אחד אל המעלה שלפניה ונכתוב על הח' ז' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| We lower one of these to the rank of the primes; 9 remains in the first [rank]. We write it above the zero, and the one that we lowered becomes sixty in the rank of the primes.
|
והאחד שהורדנו יהיה עשרה בראשונה נוריד מהם אל מעלת הראשונים אחד וישארו ט' בראשונה ונכתבם על הגלגל והאחד שהורדנו יהיה ששים במדרגת הראשונים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| We subtract 50 from them; 10 remains. We write [it] in the row of the result in the rank of the primes.
|
נגרע מהם נ' נשארו י' ונכתוב בטור העולה במדרגת הראשונים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| We subtract from 9 what corresponds to it in the bottom row; 6 corresponds to it. We subtract it from 9; 3 remains. We write it in the row of the result, in the first rank of the integers.
|
נשוב לגרוע מט' מה שכנגדו בטור התחתון והנה כנגדו ו' נגרע אותם מט' נשארו ג' ונכתב אותם בטור העולה במדרגה הראשונה מהשלמים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| We also subtract from 7 what corresponds to it in the bottom row, but there is nothing corresponding to it. So, we write 7 in the row of the result in its appropriate place.
|
נשוב לגרוע מז' מה שכנגדו בטור התחתון והנה אין כנגדו דבר ולזה נכתב ז' בטור העולה במקומם הראוי להם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Next we subtract 2 that is in the bottom row from what corresponds to it in the upper row, but there is only a zero corresponding to it. We cannot subtract 2 from a zero. In the rank next to it there is 1. We take the one that is in the rank next to it and write a zero instead of it, since nothing remains in that rank.
|
נשוב לגרוע ב' אשר בטור התחתון ממה שכנגדם בטור העליון והנה אין כנגדם כי אם גלגל ולא נוכל לגרוע ב' מגלגל ובמעלה השנית לה א'[536] נקח האחד אשר במעלה הסמוכה לה ונכתב במקומו גלגל לפי שלא ישאר במדרגה ההיא דבר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| When we lower the one we took to the preceding rank, it becomes ten. We subtract from it the 2 that is in the bottom row; 8 remains. We write it in its appropriate place in the third rank of the row of the result.
|
ויהיה האחד אשר לקחנו כשנורידהו אל המעלה אשר לפניה עשרה נגרע מהם ב' אשר בטור התחתון ונשארו ח' ונכתב אותם במקומם הראוי להם בטור העולה במדרגה השלישית[537] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| We subtract from the zero what corresponds to it in the bottom row, but there is nothing there, so we write a zero in the row of the result, in the fourth rank.
|
נשוב לגרוע מהגלגל מה שכנגדו בטור השפל ואין שם דבר ונכתב גלגל בטור העולה במדרגה הרביעית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Then, we subtract from 3 what corresponds to it in the bottom row, but there is nothing there, so we write it in the row of the result, in the fifth rank.
|
נשוב לגרוע מג' מה שכנגדו בטור התחתון ואין שם בו דבר[538] ונשאר ג' ונכתבם בטור העולה במעלה החמישית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Therefore, the result is thirty thousand, eight hundred and seventy-three integers, 10 primes, 45 seconds, 58 thirds, 47 fourths, 53 sixths. | והנה העולה הוא שלשים אלף ושמנה מאות ושבעים ושלשה שלמים י' ראשונים מ"ה שניים נ"ח שלישיים מ"ז רביעיים כ"ג ששיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Deduce from this. | והקש על זה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Sometimes the calculation in astronomy brings you to subtract a larger number from a smaller one, this happens in the motions of the stars. | ופעמים יביאך החשבון בחכמת התכונה לגרוע מספר [539]רב ממספר מעט וזה במהלכות הכוכבים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| So, you add the degrees of the circle, which are three hundred and sixty, to the smaller number you want to subtract from, and then you can subtract whatever you want. | ואז תוסיף מעלות הגלגל שהם שלש מאות וששים על המספר המעט שבאת לגרוע ממנו ותוכל לגרוע מה שתרצה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| As there is no number that is greater than three hundred and sixty in the positions of the stars. | לפי שאין להם מספר משלימים במקומות הכוכבים מוסיף על שלש מאות וששים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Because when a number is greater than three hundred and sixty, they [= the astronomers] subtract [360] and take only the remainder. | כי כאשר היה להם יותר משלש מאות וששים ישליכום ויקחו הנשאר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Likewise, the common calculation of the new moon brings you to subtract a larger number from a smaller number. | ובזה יביאך החשבון בחשבון מולד הלבנה המתפשט בהמון לגרוע ממספר מעט מספר רב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| So, you add 7 days to the smaller number, and then you can subtract whatever you like. | ואז תוסיף על המספר המעט ז' ימים ותוכל לגרוע מה שתרצה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The reason is that when those who calculate the new moon have a number greater than 7 days, they subtract the 7 days from it and keep only the remainder. | וסבת זה כי מחשבי המולדות כאשר יהיה להם מספר מוסיף על ז' ימים ישליכו ממנו ז' ימים ויקחו הנשאר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Deduce from this. | והקש על זה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Chapter Two on the Addition of Equal Numbers, which is the Multiplication of a Number by a Number |
השער השני בחבור מספרים דומים והוא הכאת מספר על מספר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| You already know that when there are four proportional numbers, meaning that the ratio of the first to the second is as the ratio of the third to the fourth, the product of the first to the fourth is as the product of the second to the third.
|
כבר ידעת שכאשר היו ארבעה מספרים מתיחסים רצוני שיחס הראשון אל השני כיחס השלישי אל הרביעי הנה שטח הראשון ברביעי כמו שטח השני בשלישי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Hence it is clear that the product of a unit of the second [rank] by a unit of the fourth [rank] is a unit of the fifth [rank].
|
ולזה יתבאר שהכאת אחד מהשנית באחד מהרביעית הוא אחד מהחמישית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| For, the ratio of a unit of the first [rank] to a unit of the second [rank] is as the ratio of a unit of the fourth [rank] to a unit of the fifth [rank].
|
וזה שיחס אחד מהראשונה אל אחד מהשנית כיחס אחד מהרביעית אל אחד מהחמישית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Thus, the product of a unit of the second [rank] by a unit of the fourth [rank] is as the product of a unit of the first [rank] by a unit of the fifth [rank].
|
אם כן שטח אחד מהשנית באחד מהרביעית הוא כמו שטח אחד מהראשונה באחד מהחמישית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| But, the product of a unit of the first [rank] by a unit of the fifth [rank] is a unit of the fifth [rank], since it is one time the unit of the fifth [rank].
|
אבל שטח אחד מהראשונה באחד מהחמישית הוא אחד מהחמישית לפי שהוא אחד מהחמישית פעם אחת | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Therefore, the product of a unit of the second [rank] by a unit of the fourth [rank] is a unit of the fifth [rank].
|
אם כן שטח אחד מהשנית באחד מהרביעית הוא אחד מהחמישית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Likewise, it is clear that the product of 1 of the third [rank] by 1 of the fourth [rank] is 1 of the sixth [rank].
|
וגם יתבאר שהכאת א' מהשלישית בא' מהרביעית הוא א' מהששית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Since, according to the equivalence ratio, the ratio of a unit of the first [rank] to a unit of the third [rank] is equal to the ratio of a unit of the fourth [rank] to a unit of the sixth [rank].
|
לפי שביחס השווי יהיה יחס[540] אחד מהראשונה אל אחד מהשלישית כיחס אחד מהרביעית אל אחד מהששית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| When you apply that, it becomes clear to you that for any unit of any rank, which is multiplied by a unit of any rank, [the unit of the product] is a unit of the rank, whose distance from the unit of the multiplicand is as far backwards as the distance of the rank of the unit of the multiplier [from the first rank], which is equal to the sum of the ranks of the multiplier and the multiplicand minus one. | וכאשר תנהיג זה יתבאר לך שכל אחד ממעלה איזו שתהיה שיוכה על אחד ממעלה איזו שתהיה הוא אחד מהמעלה אשר מרחקה מהאחד המוכה לאחריה כמרחק מעלת[541] האחד המכה מהראשונה[542] וזה שוה למספר מעלת[543] המכה והמוכה [544]פחות אחת | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Because one of the ranks is counted twice. | לפי שאחת המעלות תמנה שתי פעמים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
משל זה שיחס הראשונה אל הרביעית כיחס החמישית אל השמינית כי השמינית רביעית לחמישית בהמנות החמישית וכבר נמנית החמישית במספר מעלותיה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם כן החמישית נמנית שתי פעמים ולזה תחסר אחת ממספר מעלות המקובץ | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If any number of units of any rank is multiplied by any number of units of any rank, it has already been clarified that the product must be placed in the rank whose distance from the rank of the multiplicand is as far backwards as the distance of the multiplier from the first [rank]. | ואם הוכה מספר מה מאחדי מעלה מה על מספר מה מאחדי מעלה מה בזה בעצמו יתבאר שהעולה יושם במעלה אשר מרחקה מהמעלה המוכה[545] לאחריה כמרחק המכה מהראשונה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והמשל שיהיה לנו להכות ששה מהשלישית על שבעה מהשנית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והוא מבואר שיחס אחד מהראשונה אל אחד מהשלישית כיחס אחד מהשנית אל אחד מהרביעית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אבל יחס הששה מהראשונה אל ששה מהשלישית הוא כיחס אחד מהראשונה אל אחד מהשלישית לפי שהכפלים שוים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ויחס שבעה מהשנית אל שבעה מהרביעית הוא כיחס אחד מהשנית אל אחד מהרביעית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם כן יחס ששה מהראשונה אל ששה מהשלישית כיחס שבעה מהשנית אל שבעה מהרביעית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם כן שטח ששה מהשלישית בשבעה מהשנית כמו שטח ששה מהראשונה בשבעה מהרביעית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואולם שטח אחד מהראשונה בשבעה מהרביעית הוא[546] מהרביעית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם כן שטח ששה מהראשונה בשבעה מהרביעית הוא מאחדי הרביעית וזה כי הוא ששה דמיוני שבעה מהרביעית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| By this proof itself it is clear that the product of "geometrical fractions" belongs to the rank whose distance from the rank of the multiplicand is as the distance of the multiplier from that of the rank of the integers, because these ranks are also proportional, and therefore the result of multiplication of a number from one rank of fractions by a number from one rank of fractions is of the rank whose positional number is the same as the sum of the ranks of the multiplier and the multiplicand. | ובזה המופת בעינו התבאר שהכאת שברים משברי חכמי התכונה בשברים הם מהמעלה אשר מרחקה מהמוכה לפניה כמרחק המכה ממעלות האחדים השלמים לפי שהמדרגות ההם הם מתיחסות גם כן ולזה יהיה העולה מהכאת מספר ממדרגת[547] שברים על מספר ממדרגת[548] שברים מהמדרגה אשר מספר מעלותיה כמספר מעלות המכה והמוכה מקובצים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The reason for this is that the rank of the units from which the fractions start is not counted [in the number of their ranks], but the counting of the ranks starts from the primes. This is very clear. | והסבה בזה שלא נמנות בשברים מדרגת האחדים אשר ממנה התחלתם אך התחלת מנין המעלות מהשברים הראשונים וזה מבואר [549]מאד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| It is clear from this that the result of multiplying units of the first rank by fractions of any rank belongs to the rank of the fractions themselves. | וכזה התבאר מזה שהעולה בהכאת האחדים מהמעלה הראשונה על השברים ממעלה מה הוא ממעלת השברים בעצמה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| This is what we wanted to explain, therefore what we wanted to explain is clarified by this. | וזה מה שראינו להציע הנה יתבארו בו[550] מה שנרצה לבארו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| However, the multiplication of fractions by fractions or integers is actually division, so we will not explain it in this chapter. | ואולם הכאת השברים בשברים או בשלמים הוא חלוק על דרך האמת ולזה לא נבאר ענינו בזה השער | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The way you should apply when multiplying a number by a number: | הדרך אשר תלך בה בהכאת מספר על מספר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| You should write down the multiplier in a row according to its ranks, and the multiplicand in a row below it, also according to its ranks. | ראוי לך שתכתוב המכה בטור אחד כפי מעלותיו והמוכה בטור אחד תחתיו כפי מעלותיו גם כן | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| To make it easier for you, write the number that has fewer ranks in the first row, even if they have greater numerical values, for it all leads to the same thing, meaning the multiplication of the one by the other is the same as the multiplication of the other by the former [= the order of the factors does not matter]; this is proved by Euclid. | ולהקל מעליך שים המספר אשר יותר מעט במעלות אחז בטור הראשון ואם הוא רב בכמות כי הכל הולך אל מקום אחד רצוני שהכאת האחד באחר כמו הכאת האחר בו וזה התבאר[551] מאקלידיס | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Then, create as many rows under these two rows as the number of ranks, in which there is a number, in the first row. These should be the rows in which you should write the result of the [individual] multiplications of the numbers by each other. | אחר כן תעשה טורים תחת אלו שני הטורים כמספר המעלות אשר יש בהם מספר בטור הראשון והם יהיו[552] הטורים אשר תכתוב בהם העולה בהכות אלו המספרים איש אל אחיו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| After you have done that, multiply the first number of the upper row by the first number of the lower row, and write the result in the first of the result rows, in the corresponding rank, according to what preceded. | ואחר עשותך זה הכה המספר הראשון שבטור העליון על המספר הראשון שבטור התחתון והעולה תשים בראשון מטורי העולה במעלה הראויה לפי מה שקדם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| To make it easier for you, so that you do not have to calculate where to write the result each time, multiply the first number of the upper row by what is in the first rank of the bottom row, and write the result in the first result row, in the rank of the multiplier. | ולהקל מעליך שלא תצטרך לחשוב אנה[553] תשים העולה כפעם בפעם הכה המספר הראשון שבטור העליון על מה שבמדרגה הראשונה שבטור התחתון וכתוב העולה בטור הראשון מטורי[554] העולה במדרגת המכה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Then, multiply it again by what is in the second rank of the bottom row, and write the result in the rank that is next to the rank in which you started writing the result. | תשוב להכותו על מה שבמדרגה במעלה השנית בטור התחתון ותכתוב העולה במעלה הנמשכת למעלה שהחלות לכתוב בה[555] העולה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| And so multiply the first number of the upper row by everything that is in the ranks of the lower row, and write the result each time in the rank following the rank in which you wrote before this multiplication. | וכזה תכה המספר הראשון שבטור העליון על כל מה שבמדרגות הטור התחתון ותכתוב העולה כפעם בפעם[556] במעלה הנמשכת אחר המעלה אשר כתבת בה קודם זאת ההכאה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Then, multiply the second number of the upper row by everything that is in the ranks of the lower row successively and start writing the result in the multiplier rank, in the second of the result rows, followed by the ranks of this result row successively. | אחר כן תשוב להכות המספר השני שבטור העליון על כל מה שבמדרגות הטור התחתון על הסדר ותחל לכתוב העולה במעלת המכה בטור השני מטורי[557] העולה ואחר ימשכו מעלות הטור העולה על הסדר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Then, multiply the third number of the upper row by everything that is in the ranks of the bottom row successively, and write down the result in the order given above. | [558]אחר כן תשוב להכות המספר השלישי שבטור העליון על כל מה שבמדרגות הטור התחתון על הסדר ותכתוב העולה על הסדר שקדם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| And so on until all the numbers in the upper row are gone. | וכן עד כלות כל מספרי הטור העליון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Then, add up all the numbers of the result rows and write the result in one row below the result rows; this is the product of the first number by the other [number], because all the parts of one has been multiplied by all the parts of the other. | אחר כן תחבר כל מספרי טורי[559] העולה ותכתוב העולה בטור אחד[560] תחת טורי העולה והוא שטח המספר האחד בשני כי כבר הוכו כל[561] חלקי זה בכללם על חלקי זה בכללם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון נרצה בזאת הצורה להכות ז' אלפי אלפים ושלשים על מאה ושמנים אלף ושש מאות וארבעים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה המספר אשר החזיק ביותר מעט מהמעלות הוא ז' אלפי אלפים ושלשים ונכתבהו בטור העליון במקומותיו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והמספר האחר כתבנו בטור אחר תחתיו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה המספר הראשון שבטור העליון הוא ג' בשנית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הכינו ג' על מה שבמדרגה הראשונה מהטור התחתון שהוא גלגל ועלה גלגל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכתבנוהו במעלה השנית בראשון שבטורי[562] העולה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הכינו ג' על מה שבמדרגה השנית מהטור התחתון שהוא ד' ועלה י"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונכתב ב' במדרגה השלישית בטור העולה והעשרה יהיו אחד בשנית לה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הכינו ג' על מה שבמדרגה השלישית מהטור התחתון שהוא ו'[563] ועלה י"ח ואחד שנשאר לנו שם והנה י"ט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונכתב ט' במדרגה הרביעית בטור העולה והעשרה יהיו אחד בשנית לה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הכינו ג' על מה שבמדרגה הרביעית שהוא גלגל ועלה גלגל ואחד[564] שנשאר לנו שם והנה א' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכתבנוהו בטור העולה אחר ט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הכינו ג' על מה שבמדרגה החמישית שהוא ח' ועלה כ"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונכתב ד' אחר הא' והכ' יהיו שנים בשנית לה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הכינו ג' על מה שבמדרגה הששית שהוא א' ועלה ג' וב' שנשארו לנו שם והנה ה' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונכתב ה' אחר הד' בטור העולה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ופה נשלמה הכאת ג' על כל מה שבמדרגות הטור התחתון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונכה המספר הבא אחר הג'[565] בטור העליון שהוא ז'[566] על כל מה שבטור התחתון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הכינו ז' על גלגל ועלה גלגל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונשימהו בטורי[567] העולה בטור השני [568]במעלה השביעית כנגד ז' שהיא במעלה השביעית[569] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הכינו ז' על ד' ועלה כ"ח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונכתב ח' אחר הגלגל והכף תהיינה ב' במעלה השנית לה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הכינו ז' על ו'[570] ועלה מ"ב וב' שנשארו לנו שם והנה מ"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונכתב ד' אחר הח' והמ' תהיינה ד' בשנית לה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הכינו ז' על גלגל ועלה גלגל וד' שנשארו לנו שם והנה ד' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונכתבם אחר הד' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הכינו ז' על ח' ועלה נ"ו[571] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונכתב ו'[572] אחר הד' והנ' תהיינה ה' בשנית לה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הכינו ז' על א' ועלה ז' וה'[573] שנשארו לנו שם והנה י"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונכתב ב' אחר הו' והי'[574] נעשה א' במעלה השנית לה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ופה נשלמה הכאת כל מספרי הטור העליון על כל מספרי הטור התחתון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה העולה הוא[575] גלגל בראשונה גלגל בשנית ב' בשלישית ט' ברביעית א' בחמישית ד' בששית ה' בשביעית ה'[576] בשמינית ד' בתשיעית ד' בעשירית ו' באחד עשרה ב' בשנים עשרה א' בשלש עשרה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Deduce from this. | והקש על זה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If you want to know how much is the square of a given number: | ואם רצית לדעת כמה יעלה מספר המרובע ההווה ממספר מונח[577] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Write the number whose square you want to know in a row, then write it again in another row under this row and multiply all the digits of the upper row by everything that is in the ranks of the bottom row; you will receive the required. | תכתוב המספר אשר רצית לדעת מרובעו בטור אחד ותחתיו תשוב ותכתבהו בטור אחר תחת הטור הזה ותכה כל מספרי הטור העליון על כל מה שבמדרגות הטור השפל ויצא לך המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If you want to know how much is the cube number of a given number: | ואם רצית לדעת כמה יעלה המספר המעוקב ההווה ממספר מונח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| You need to make two diagrams: | תצטרך לעשות שתי תמונות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| First you multiply this number by itself, so you receive the square of the number whose cube you want to know. | ראשונה תכה המספר ההוא על עצמו ויצא לך מרובע המספר שרצית לדעת מעוקבו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Then, you do a second diagram and multiply the number whose cube you are looking for by its square you received; the result is the required. | עוד תעשה תמונה אחרת ותכה המספר המונח שרצית לדעת מעוקבו על מרובעו שיצא לך והעולה הוא המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| To make it easier for you, I will give you many ways by which to calculate the product of a number by a number easily. | ולהקל מעליך אתן[578] לך דרכים רבים לחשוב בהם הכאת מספר במספר בקלות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Multiplication by Rounding |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| You already know that multiplying a number in the first rank by a number in the first rank is an easy procedure and so is the multiplication of a fractional number, meaning of a number in the first and second rank by a number in the first rank. | כבר ידעת שהכאת מספר ממעלה ראשונה במספר ממעלה ראשונה הוא קל המעשה וכן הכאת מספר נשבר רצוני מספר מהמעלה הראשונה והשנייה על מספר מעלה ראשונה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If you have to multiply a fractional number by a fractional number, round one of the numbers to the nearest side. | ואם היה לך [579]להכות מספר נשבר על מספר נשבר השלים המספר האחד מהם אל הצד אשר הוא היותר[580] קרוב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If you have added to that number, to round it to the nearest unit, subtract from the other number what you added to the first number, multiply the remainder by the rounded number you have, and keep the result. | ואם הוספת על זה המספר להשלימו אל הכלל הקרוב גרע מהמספר[581] האחר כשיעור מה שהוספת על המספר הראשון והנשאר הכה אותו על המספר השלם אשר בידך ושמור העולה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If you have subtracted from the number, to round it, add to the other number what you subtracted from the first number, multiply what you are left with by the rounded number you have, and keep the result. | ואם גרעת מזה המספר להשלימו הוסף על המספר האחר כשעור מה שגרעת מזה המספר הראשון והנשאר בידך הכה אותו על המספר השלם אשר בידך ושמור העולה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Then, see how much the larger number after the addition or subtraction exceeds the smaller number before the rounding, multiply the excess by the number you added to one of the numbers, and keep the result; it is the second reserved. | אחר כן ראה[582] המספר הגדול אחר התוספת או אחר הגרעון כמה הוא מוסיף על המספר הקטן טרם התקון והתוספת ההוא ערוך על שעור המספר שהוספת על אחד מהמספרים והעולה שמור והוא השמור השני | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Then, see from which number you subtracted: | וראה[583] אחר כן מאי זה מספר גרעת | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If you have subtracted from the larger number, subtract the second reserved from the first reserved; the remainder you receive is the required. | ואם גרעת מהמספר הגדול תגרע השמור השני מהשמור הראשון והנשאר בידך הוא המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If you have added to the larger number, add the second reserved to the first reserved; this is the required. | ואם הוספת על המספר הגדול הוסף השמור השני על השמור הראשון[584] והוא המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| I shall give you examples for this: | ואתן לך על זה משלים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נרצה להכות ל"ד על נ"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
השלמנו מספר נ"ז אל הכלל הקרוב ויהיה ס' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולפי שס' מוסיף על נ"ז שלשה נגרע מל"ד שלשה ויהיו ל"א ונכה ל"א על ס' יהיו אלף ושמנה מאות וששים והוא השמור הראשון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולפי שס' מוסיף על ל"ד כ"ו נערך כ"ו על שלשה והנה ע"ח והוא השמור השני | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולפי שהוספנו על המספר הגדול נוסיף השמור השני על השמור הראשון והנה אלף ותשע מאות ול"ח והוא המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ובמשלינו זה אם הורדנו נ"ז אל הכלל שלמטה ממנו יהיה נ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הוספנו על ל"ד ז'[585] והנה מ"א ערכנו מ"א על נ' והנה אלפים וחמשים והוא השמור הראשון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומפני שנ' מוסיף על ל"ד י"ו[586] תערך י"ו על ז' והנה קי"ב והוא השמור השני | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולפי שגרענו מהמספר הגדול נגרע השמור השני מהשמור הראשון ונשאר אלף וט' מאות ול"ח והוא המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וגם כן במשלינו זה אם הורדת[587] ל"ד אל הכלל הקרוב אליו יהיה [588]ל' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הוספת על נ"ז ד' והנה ס"א ערכת ל' על ס"א והנה אלף ושמנה מאות ושלשים והוא השמור הראשון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה יתרון ס"א על ל"ד הוא כ"ז ערכנו[589] כ"ז על ד' והנה ק"ח והוא השמור השני | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולפי שהוספת על המספר הגדול תוסיף השמור[590] השני על השמור הראשון והנה אלף ותתקל"ח[591] והוא המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם העלית ל"ד אל מ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תגרע מנ"ז ו' והנה נ"א[592] תערכם על מ' והנה אלפים וארבעים והוא השמור הראשון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה יתרון נ"א על ל"ד הוא י"ז תערכם על ו' והנה[593] ק"ב והוא[594] השמור השני | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולפי שגרעת מהמספר[595] הגדול תגרע השמור השני מהשמור הראשון ונשאר בידך אלף[596] ותתקל"ח[597] והוא המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Sometimes this procedure leads you to multiply a number by itself, then this procedure is very easy for you. | ופעמים יצא לך לפי זה הדרך שיהיה לך להכות המספר על עצמו ואז יקל מאד זה הדרך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
משל זה שיהיה לך להכות מ"ג על נ"ז[598] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תשלים[599] מ"ג אל נ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תחסר מנ"ז[600] שעור ההשלמה ויהיה נ' ויהיה לך להכות נ' על נ' ולחסר מהעולה מרובע ז' והנשאר הוא המבוקש[601] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| This is very clear from what preceded at the beginning of the first section of this book, note it and you will find it. | וזה מבואר מאד[602] ממה שקדם בראש המאמר הראשון מזה הספר והבן ותמצא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Another way of doing this: round one number to the nearest unit, multiply the result by the other number and keep the result. Multiply the other number also by the round number and keep the result; it is the second reserved. | דרך אחרת בזה השלים המספר האחד אל הכלל הקרוב ועל העולה ערוך המספר האחר[603] ושמור העולה גם ערוך המספר האחר על שעור ההשלמה[604] ושמור העולה והוא השמור השני | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If the rounding was done by addition, then we subtract the second reserved from the first reserved; the remainder is the required. | ואם היתה ההשלמה לתוספת נגרע השמור השני מהשמור הראשון והנשאר הוא המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| But, if the rounding was done by subtraction, then add the second reserved to the first reserved; the result is the required. | ואם היתה ההשלמה למגרעת תוסיף השמור השני על השמור הראשון והעולה הוא המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The reason for this is easily deduced from the second [proposition] of the first section. | והסבה בזה תצא מב' מהמאמר הראשון בקלות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה במשלינו הקודם השלמנו נ"ז[605] אל הכלל הקרוב והנה ס' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ערכנו ס' על ל"ד והנה אלפים וארבעים והוא השמור הראשון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה שעור ההשלמה הוא שלשה ערכנו שלשה על ל"ד והנה ק"ב והוא השמור השני | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולפי שההשלמה היתה לתוספת נגרע השמור השני מהשמור הראשון ונשאר אלף ותתקל"ח והוא המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וגם כן במשלינו זה אם השלמנו ל"ד אל הכלל הקרוב יהיו ל' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ערכנו ל'[606] על נ"ז [607]עלה אלף ת"ש[608] ועשר והוא השמור הראשון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה שעור ההשלמה הוא ד' ערכנו ד' על נ"ז ועלה רכ"ח[609] והוא השמור השני | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולפי שההשלמה היתה למגרעת נוסיף השמור השני על השמור הראשון ויעלה אלף ותתקל"ח והוא[610] המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Squaring |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If you want to know the square of a given "fractional" number: round the number to the nearest unit, subtract the rounding number from the given number, multiply the remainder by the rounded number, and add the square of the rounding number to the result; it is the required. | ואם רצית לדעת מרובע מספר נשבר מונח הנה תשלים המספר אל הכלל הקרוב וכשעור ההשלמה תגרע מהמספר המונח והנשאר תכה על המספר המושלם והוסף על העולה מרובע מספר ההשלמה והנה המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
משל זה אם רצית לדעת מרובע מ"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה הכלל הקרוב הוא נ' ומרחקו ממ"ז הוא שלשה תגרעם ממ"ז והנה מ"ד ערכת[611] מ"ד על נ' והנה אלפים ומאתים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה המרחק הוא שלשה שהוא תשעה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה אלפים ור"ט[612] והוא המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Another way to easily find the square of a number of one rank: see the ratio of that number to the unit of the next rank, take the part determined by this ratio from the number you want to square and multiply it by the unit of the next rank, and this is the required. | דרך לדעת בקלות מרובע מספר ממעלה אחת ראה יחס המספר אל אחד מהמעלה הנמשכת וקח כמו היחס ההוא מהמספר[613] שרצית לדעת מרובעו וערכהו על אחת מהמעלה הנמשכת והוא[614] המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון רצינו לדעת כמה מרובע שלשים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה יחס שלשים אל מאה שהוא אחד מהמעלה הנמשכת הוא שלש עשיריותיו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
קח שלש עשיריות שלשים והנה ט' ערכם על מאה והנה ט' מאות והוא[615] המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והיה זה כן[616] לפי ששלשים הוא אמצעי ביחס בין ט' ובין ק' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If the number you want to square consists of two successive ranks, round the number to the nearest unit, find its square and keep the result. | ואם היה המספר שרצית לדעת מרובעו משתי מעלות נמשכות השלים המספר אל הכלל הקרוב ותדע מרובעו ושמור העולה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Then, add the rounded number to the fractional number and multiply it by the rounding number, and it is the second reserved. | אחר כן חבר המספר השלם אם[617] המספר הנשבר וערכהו על שעור ההשלמה והוא יהיה השמור השני | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If the rounding was done by addition, subtract the second reserved from the first reserved. | ואם היתה ההשלמה לתוספת תגרע השמור השני מהשמור הראשון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If the rounding was done by subtraction, add the second reserved to the first reserved; the result is the required. | ואם היתה ההשלמה[618] לגרעון תוסיף השמור השני על השמור הראשון והעולה הוא המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה אם רצית לדעת מרובע שלשים ושלשה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה תשלים המספר אל הכלל הקרוב והוא[619] ל' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומרובע ל' הוא ט' מאות והוא השמור [620]הראשון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אחר זה תחבר שלשים עם שלשים ושלשה והנה ס"ג ערכם על[621] ג' שהוא שעור ההשלמה והנה[622] קפ"ט והוא השמור השני | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולפי שההשלמה היתה לגרעון נוסיף[623] קפ"ט על השמור הראשון והנה אלף ופ"ט והוא הדרוש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם השלמת ל"ג אל מ' במשלינו זה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה יהיה מרובעו י"ו מאות ושמור | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותחבר ל"ג עם מ' והנה ע"ג תערכם על[624] ז' והנה[625] תקי"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולפי שההשלמה היתה לתוספת תגרע[626] תקי"א מהשמור הראשון וישאר לך[627] אלף ופ"ט והוא הדרוש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והיה זה כן לפי שיתרון מרובע מ' על מרובע ל"ג הוא כמו שטח ז' במ' ושטח ז' בל"ג וזה[628] שוה לשטח ז' בע"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Deduce from this. | והקש על זה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Another way: take a third of the number whose square you are looking for, take its square and keep it. Then, raise it to the next rank and subtract the reserved from the result; the remainder is the required. | דרך אחרת קח שלישית המספר שרצית לדעת מרובעו קח מרובעו ושמור אחר כן העלהו אל המעלה הנמשכת וגרע מהעולה השמור והנשאר[629] הוא[630] המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון רצית לדעת מרובע ל"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
לקחנו שלישיתו והוא[631] י"א ומרובעו קכ"א העלינו קכ"א אל המעלות הנמשכות והנה אלף ומאתים ועשר גרענו מהם קכ"א וישאר אלף ופ"ט והוא המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והיה זה כן לפי שאלף ומאתים ועשר הוא[632] עשרה דמיוני מספר קכ"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה יחס מרובע ל"ג אל מרובע י"א הוא יחס צלעו אל צלעו שנוי ביחס אבל יחס צלעו אל צלעו שנוי ביחס הוא יחס תשעה אל אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם כן מרובע ל"ג הוא כמו תשעה דמיוני מרובע י"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכבר היה אלף ור"י עשרה דמיוני מרובע י"א[633] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
א"כ כאשר גרענו[634] מאלף ומאתים ועשר מרובע י"א[635] היה הנשאר שוה למרובע ל"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Deduce from this. | והקש על זה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Chapter Three – Summing Successive or Proportional Numbers |
השער השלישי בחבור מספרים נמשכים או מתיחסים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם רצית לחבר מספרים נמשכים מן האחד עד מספר מונח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
קח חצי מרובע המספר המונח וחברהו עם[636] חצי המספר המונח והוא[637] המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון אם רצית לחבר אחד ושנים ושלשה וארבעה וכן עד עשרה ועשרה עמהם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
קח חצי מרובע עשרה וחציו והנה נ"ה וככה המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
[638]דרך אחרת ערוך המספר ההוא על חצי המספר הנמשך לו לאחריו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
או חצי המספר ההוא על המספר הנמשך לו לאחריו והוא[639] המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה במשלינו זה תערוך י' על חצי י"א או חצי י' על י"א והנה נ"ה[640] וככה המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם היו המספרים הנמשכים בזולת דרך המספר ר"ל שהיה הראשון מספר מונח והיה השני שני דמיוני המספר המונח והשלישי שלשה דמיוניו[641] וכן בזה הדרך עד מספר מה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תחבר הנמשכים עד המספר ההוא בדרך הקודמת והעולה תערוך על המספר הראשון המונח וככה המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה שיהיה הראשון שבעה והשני ארבעה עשר והשלישי כ"א והרביעי כ"ח וימשכו בזה הדרך עד תשעה מספרים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כבר ידעת כי הנמשכים מן האחד עד תשעה הם מ"ה ערכם על[642] ז' שהוא הראשון והנה שט"ו והוא[643] המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ויהיה זה כן לפי שיחס אחד אל הראשון כיחס שנים אל השני וכיחס שלשה אל השלישי וכיחס ארבעה אל הרביעי וכיחס חמשה אל החמישי וכיחס ששה אל הששי וכיחס שבעה אל השביעי וכיחס שמנה אל השמיני וכיחס תשעה אל התשיעי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אבל יחס האחד אל קרובו כיחס הכל אל הכל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם כן יחס אחד אל שבעה שהוא הראשון כיחס הכל אל הכל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אבל שבעה ימנהו אחד כמספר אחדי שבעה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם כן כלל כל אלו המספרים ימנהו מ"ה כמספר אחדי שבעה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם כן כבר יוכה מספר[644] מ"ה בשבעה ויהיה העולה שוה לאלו המספרים מקובצים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Deduce from this. | והקש על זה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Sum of Odd Numbers |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם רצית לחבר הנפרדים הנמשכים מתחילים מן האחד עד מספר מונח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
קח מרובע המספר[645] האמצעי בין האחד והמספר המונח והנה המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה אם רצית לחבר הנפרדים הנמשכים עד תשעה והאחד עמהם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה המספר האמצעי בין תשעה ואחד הוא חמשה קח מרובעו והוא[646] כ"ה וככה המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Sum of Even Numbers |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם רצית לחבר הזוגות הנמשכים [647]עד מספר מה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה יהיה המספר הראשון שנים והשני שני דמיוניו והשלישי שלשה דמיוניו וכן ימשכו נמשכים בזולת דרך המספר וכבר קדם דרכו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולזה תקח חצי המספר האחרון לפי שהוא כמספר מספרי הזוגות הנחברים ותדע מה יעלו הנמשכים מן האחד עדיו ותערוך העולה על שנים שהוא הראשון והנה המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה אם רצית לחבר הזוגות עד עשרה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כבר ידעת שהנמשכים עד חמשה הם ט"ו ערכת אותם על שנים שהוא הראשון והנה ל' וככה המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Sum of Square Numbers |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם רצית לחבר מרובעי מספרים נמשכים מן האחד עד מספר מונח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
קח המספר המונח פחות שלישית המספר הנמשך לפניו וערכהו על נקבץ הנמשכים מן האחד עד המספר המונח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה אם רצית לדעת מרובעי המספרים[648] הנמשכים עד חמשה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה המספר הנמשך לחמשה לפניו הוא ארבעה גרענו ממנו שלישית ארבעה שהוא ד' שלישיות ונשארו ארבעה פחות שלישית ערכנום על[649] ט"ו שהוא נקבץ הנמשכים עד חמשה ועלה נ"ה וככה המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם רצית לחבר מרובעי הנפרדים הנמשכים מן האחד או מרובעי הזוגות הנמשכים עד מספר מונח רצוני שיהיה המספר המונח הוא האחרון[650] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
קח נקבץ הנמשכים עד המספר הנמשך אחר המספר המונח וערכהו על שלישית המספר המונח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה רצינו לדעת מרובעי הנפרדים הנמשכים עד תשעה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה נקבץ הנמשכים מן האחד עד עשרה הוא נ"ה ערכנום על שלישית תשעה והנה קס"ה וככה המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם רצית לחבר מרובעי מספרים נמשכים בזולת דרך המספר עד מספר מונח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ערוך מרובעי המספרים הנמשכים מן האחד עד המספר המונח על מרובע[651] המספר הראשון והעולה הוא המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה אם רצית לחבר מרובעי מספרים נמשכים[652] [653]שהראשון ארבעה והשני שמנה והשלישי שנים עשר וימשכו בזה הדרך עד שבעה מספרים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה כבר ידעת שמרובעי כל המספרים עד שבעה הם ק"מ ערכת אותם על י"ו שהוא מרובע המספר הראשון והנה אלפים ר"מ וככה המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והיה זה כן לפי שיחס אחד אל הראשון הוא כיחס שנים אל השני וכיחס כל אחד אל גילו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם כן יהיה יחס מרובע אחד אל מרובע הראשון[654] כיחס מרובע כל אחד מהם אל מרובע[655] גילו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולזה יהיה יחס מרובע האחד אל מרובע גילו כיחס מרובעי הכל אל מרובעי הכל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Sum of Cube Numbers |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם רצית לחבר מעוקבי מספרים נמשכים מן האחד עד מספר מונח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
קח מרובע נקבץ הנמשכים מן האחד עד המספר המונח והעולה הוא המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה רצינו לדעת מעוקבי[656] המספרים הנמשכים מן האחד עד ששה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה נקבץ הנמשכים מן האחד[657] עד ששה הוא כ"א לקחנו מרובעו והנה תמ"א והוא[658] המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם היו המספרים נמשכים בזולת דרך המספר עד מספר מונח ורצית לדעת מעוקביהם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תוציא מעוקבי המספרים הנמשכים מן האחד עד המספר המונח ותערוך העולה על מעוקב המספר הראשון והוא[659] המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכבר התבארה סבת זה במה שקדם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה שיהיה הראשון[660] ארבעה והשני שמנה ונמשכו בזה הדרך עד חמשה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כבר ידענו שמעוקבי המספרים הנמשכים עד חמשה הם רכ"ה ערכנום על ס"ד שהוא מעוקב המספר הראשון ועלה י"ד אלפים וד' מאות וככה המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם רצית לדעת מעוקבי הזוגות ממספרים נמשכים מן האחד עד מספר מונח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
קח מעוקבי המספרים הנמשכים עד חצי המספר המונח וערוך העולה על מעוקב המספר הראשון שהוא שנים ומעוקבו שמנה וככה המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכבר התבארה סבת זה במה שקדם רצוני שאלו המספרים הם שבים אל המספרים הנמשכים בזולת דרך המספר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה[661] אם [662]רצית לדעת מעוקבי הזוגות הנמשכים עד ששה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה מעוקבי המספרים הנמשכים עד שלשה הם[663] ל"ו[664] ערכנום על שמנה שהוא מעוקב המספר הראשון ועלה רפ"ח וככה המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומזה תוכל לדעת מעוקבי הנפרדים הנמשכים מן האחד עד מספר מונח רצוני שתדע תחלה מעוקבי כל המספרים עד המספר המונח ותוציא מהעולה מעוקבי הזוגות והנשאר הוא מעוקבי הנפרדים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
The First Term is Not One |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם רצית לחבר מספרים נמשכים בדרך המספר בלתי מתחילים מן האחד אבל יתחילו ממספר מונח ויכלו במספר מונח שני | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
קח נקבץ הנמשכים מן האחד עד המספר המונח השני ושמור וגרע מהשמור נקבץ הנמשכים מן האחד עד המספר הנמשך לפני המספר הראשון המונח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה שיהיה המספר הראשון ששה ורצינו לחבר הנמשכים לו לאחריו עד מספר אחד עשר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה נקבץ הנמשכים מן האחד עד אחד עשר הוא ס"ו גרענו ממנו מספר הנמשכים מן האחד עד חמשה שהוא ט"ו ונשארו נ"א וככה המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן תעשה במרובעי המספרים הנמשכים כאשר לא יתחילו מן האחד או במעוקביהם והסבה בזה מבוארת | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן תעשה במרובעי הנפרדים הנמשכים כאשר לא יתחילו מן האחד או במרובעי הזוגות כאשר לא יתחילו משנים לזאת הסבה בעינה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם רצית לחבר מספר מונח ממספרים נמשכים בזולת דרך המספר והיה הראשון בלתי שוה למספר ההמשך אבל הוא פחות ממנו או יתר עליו מספר מונח שני | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ערוך המספר המונח השני על המספר המונח הראשון והעולה הוא השמור הראשון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
גם כן קח נקבץ הנמשכים מן האחד עד המספר המונח הראשון וערכהו על מספר ההמשך והוא השמור השני[665] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם היה המספר הראשון מוסיף על מספר ההמשך תוסיף השמור הראשון על השמור השני | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם היה הראשון פחות ממספר ההמשך תוציא השמור הראשון מהשמור השני והנשאר הוא המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
[666]דמיון זה אם רצית לחבר שבעה מספרים שכל אחד מהם מוסיף על המספר שלפניו שלשה והראשון פחות שנים משלשה או מוסיף שנים על שלשה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה שטח שנים בשבעה הוא י"ד והוא השמור הראשון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונקבץ הנמשכים עד שבעה הוא כ"ח ערכנום על שלשה והנה פ"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם היה הראשון פחות שנים משלשה תגרע י"ד מפ"ד וישאר ע' והוא המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והסבה שאם הוספנו חסרון הראשון מהשלשה על כל אחד מהמספרים היו נמשכים מיחס שלשה ולזה נגרע מהעולה שטח שנים בשבעה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם היה הראשון מוסיף על שלשה שנים נוסיף י"ד על פ"ד ויהיו צ"ח והוא המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והסבה שאם גרענו תוספת הראשון על שלשה מכל אחד מהמספרים היו נמשכים בזולת דרך המספר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והקש על זה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם רצית לחבר מרובעי מספר מונח ממספרים נמשכים בזולת דרך המספר אלא שהמספר הראשון מתחלף ממספר ההמשך בשעור מספר מונח שני | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ערוך המונח השני על כפל נקבץ אלו המספרים והעולה תחבר עם שטח המספר המונח הראשון במרובע המספר המונח השני אם היה הראשון פחות ממספר ההמשך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם היה הראשון מוסיף על מספר ההמשך תגרע זה השטח שזכרנו מהעולה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומה שישאר בידך אחר התוספת או הגרעון הוא השמור הראשון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אחר כן קח מרובעי המספרים הנמשכים[667] מן האחד עד המספר המונח הראשון וערוך העולה על מרובע מספר ההמשך והוא השמור השני | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם היה המספר הראשון פחות ממספר ההמשך גרע השמור הראשון מהשמור השני והנשאר הוא המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם היה המספר הראשון[668] מוסיף על המספר המונח השני הוסף השמור הראשון על השמור השני והוא[669]המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה אם רצית לחבר מרובעי ז' מספרים שכל אחד מהם מוסיף על המספר שלפניו ג' והראשון מהם מוסיף שנים על [670]שלשה או פחות שנים ממנו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ויהיה תחלה הראשון מוסיף שנים על שלשה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה ידענו שנקבץ אלו המספרים מאשר לפניה הוא צ"ח וכפלהו והנה קצ"ו ערכנום על שנים והנה שצ"ב ואולם שטח מרובע שנים שהוא ארבעה בשבעה הוא כ"ח גרענום משצ"ב והנה שס"ד[671] והוא השמור הראשון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
או אם תערוך[672] שנים על כפל[673] נקבץ[674] שבעה מספרים נמשכים בזולת דרך המספר שכל אחד מהם מוסיף שלשה על שלפניו והראשון שלשה ותחבר עם העולה שטח מרובע שנים בשבעה יעלה שס"ד גם כן | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה ששטח כפל שנים בפ"ד הוא של"ו חברנו עמו שטח ארבעה בשבעה שהוא כ"ח ועלה שס"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה אלו שתי הדרכים יביאוך אל מספר אחד בעינו וזה מבואר מראש המאמר הראשון עם מעט עיון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואולם מרובעי המספרים הנמשכים עד שבעה הם ק"מ ערכנום על תשעה שהוא מרובע מספר ההמשך והנה אלף ור"ס | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חברנום עם שס"ד והנה אלף ותרכ"ד והוא המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ויהיה גם כן[675] הראשון פחות שנים משלשה במשלינו זה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה ידענו שנקבץ המספרים[676] האלו הוא[677] ע' כפלנוהו והנה ק"מ ערכנום על שנים והנה ר"פ ואולם שטח מרובע שנים בשבעה הוא כ"ח חברנום עם ר"פ והנה ש"ח והוא השמור הראשון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואולם מרובעי המספרים הנמשכים עד שבעה הם ק"מ ערכנום על ט' שהוא מרובע מספר ההמשך והנה אלף ור"ס | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
גרענו מהם ש"ח והנה תתקנ"ב[678] והוא המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והיה זה כן לפי שאם הוסף שנים על כל אחד מהמספרים היו נמשכים בזולת דרך המספר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואולם יתרון מרובע כל מספר מהם כאשר חובר עם שנים על מרובעו הוא כפל שטח שנים במספרו ומרובע שנים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר חובר זה התוספת מכל אלו המספרים היה כמו כפל שטח שנים בכל אלו המספרים נחברים וכמו שטח מרובע שנים במספר המספרים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
גרענו העולה ממרובעי המספרים הנמשכים בזולת דרך המספר בשעור זה ההמשך עד המספר המונח הראשון ונשאר המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ובזה התבאר מקביל זה עם מעט [679]עיון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Understand, and you will find it. | והבין ותמצא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם רצית לחבר מעוקבי מספר מונח ממספרים נמשכים בזולת דרך המספר אלא שהמספר הראשון מתחלף למספר ההמשך בשעור מספר מונח שני | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה אם היה המספר הראשון פחות ממספר ההמשך[680] תקח מרובעי אלו המספרים ותערוך העולה על שלשה דמיוני המספר המונח השני ותשמור | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד תערוך שלשה מרובעי המספר המונח השני על נקבץ אלו המספרים ושמור | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד תערוך מעוקב המספר המונח השני על המספר המונח הראשון ותחבר העולה עם שני השמורים ויהיה[681] בידך השמור הראשון המתוקן | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אחר כן קח מעוקבי המספרים הנמשכים מן האחד עד[682] המספר המונח הראשון וערכם על מעוקב מספר ההמשך ומהעולה גרע השמור הראשון המתוקן והנשאר הוא המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה אם רצית לחבר מעוקבי שבעה מספרים שכל אחד מהם מוסיף על שלפניו שלשה והראשון פחות משלשה שנים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה כבר ידעת שמרובעי אלו המספרים הם תתקנ"ב ערכנו זה על שלשה דמיוני שנים שהוא ו' והנה ה' אלפים ותשי"ב ונשמרם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וגם כן הנה נקבץ אלו המספרים הוא ע' ערכנום על שלשה דמיוני מרובע שנים שהוא י"ב והנה תת"מ ונשמרם גם כן | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ערכנו מעוקב שנים שהוא שמנה על ז' והנה נ"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חברנום[683] עם שני השמורים והנה ו' אלפים ותר"ח והוא השמור הראשון המתוקן | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הוצאנו מעוקבי המספרים הנמשכים מן האחד עד ז' והנה תשפ"ד ערכנום על כ"ז שהוא מעוקב שלשה והנה כ"א אלפים וקס"ח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
גרענו מהם השמור הראשון המתוקן ונשאר י"ד אלפים ותק"ס[684] והוא המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם היה המספר הראשון מוסיף על מספר ההמשך מספר מונח שני הוצא נקבץ המספר המונח הראשון ממספרים[685] נמשכים בזולת דרך המספר בשעור זה ההמשך המונח וכבר ידעת אופן זה המעשה במה שקדם ערוך [686]העולה על שלשה דמיוני מרובע המונח השני ושמור העולה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
גם קח מרובעי המספר המונח הראשון ממספרים נמשכים בזולת דרך המספר בשעור זה ההמשך המונח וערוך העולה על שלשה דמיוני המספר המונח השני ושמור העולה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד תערוך מעוקב המספר המונח השני על המספר המונח הראשון וחבר העולה עם שני[687] השמורים והעולה יהיה השמור הראשון המתוקן | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אחר כן הוצא מעוקבי המספר המונח הראשון ממספרים נמשכים בזולת דרך המספר בשעור ההמשך המונח וחבר עם העולה השמור הראשון המתוקן והנה המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה במשלינו הקודם רצוני שיהיה המספר הראשון מוסף שנים על שעור ההמשך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה ידענו כי מרובעי שבעה מספרים נמשכים בהמשך שלשה שלשה הם אלף ור"ס ערכנו אלף ור"ס על שלשה דמיוני המספר המונח השני שהוא ו' ועלה ז' אלפים ותק"ס[688] ונשמור העולה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וגם כן הנה נקבץ המספרים[689] הנמשכים בהמשך שלשה עד שבעה הוא פ"ד ערכנום על שלשה דמיוני מרובע המספר המונח השני שהוא י"ב ועלה אלף וח' ונשמרם גם כן | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וגם כן הנה מעוקב המספר המונח השני הוא ח' ערכנוהו[690] על המספר המונח הראשון שהוא ז' ועלה נ"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חברנום עם שני השמורים ועלה ח' אלפים ותרכ"ד והוא השמור הראשון המתוקן | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וגם כן הנה מעוקבי שבעה מספרים נמשכים בהמשך שלשה הוא כ"א אלפים וקס"ח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חברנום עם השמור הראשון המתוקן ועלה כ"ט אלפים ותשצ"ב[691] והוא המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והיה זה כן שאם נגרע מכל[692] אחד מאלו המספרים שנים היו נמשכים בהמשך שלשה ואולם מעוקב כל מספר מהם פחות ממעוקב המספר כשהוסף עליו שנים כמו שלשה דמיוני שטח[693] מרובע המספר ההוא על שנים וכמו שלשה דמיוני שטח[694] המספר ההוא[695] על מרובע שנים וכמו מעוקב שנים וכאשר קבצנו זה בכל המספרים היה מה שזכרנו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותבין ותמצא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ובזה [696]התבארה הסבה במקביל זה עם מעט עיון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Finding the Last Term |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם רצית לדעת מה יעלה המספר האחרון ממספר מונח ממספרים מתיחסים על יחס מונח מתחילים מן האחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
קח מרובע היחס והנה השלישי קח מרובע השלישי והנה החמישי קח מרובע החמישי והנה התשיעי ובזה הדרך תוכל לדעת ממרובע כל מספר מהם מספר קצהו וכאשר תגיע אל מספר קרוב מהמספר המונח שמור מספרו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אחר כן תדע כמה מרחקו מהמספר המונח[697] גם דע מה יעלה המספר מאלו המתיחסים[698] אשר מרחקו ככה מן האחד ועל מספרו תערוך השמור והנה המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
משל זה אם רצית לדעת מה יעלה המספר האחרון מחמשה עשר מספרים מתיחסים על יחס[699] מונח מתחילים מן האחד והיה היחס המונח שלשה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ובזה יהיה המספר השני שלשה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
לקחנו מרובע ג' שהוא ט' והנה השלישי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
לקחנו מרובע ט' שהוא פ"א והנה החמישי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
לקחנו מרובע פ"א שהוא ו' אלפים ותקס"א והנה התשיעי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם נקח מרובע התשיעי יהיה לנו השבעה עשר ויעבור המספר המונח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|
ולזה נראה כמה מרחק התשיעי מן החמש עשרה והנה החמש עשרה הוא השביעי לו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולזה ראוי שנדע כמה מספר המספר השביעי לאחד וידענו כי המספר החמישי לאחד הוא פ"א והשביעי הוא שלישי לחמשי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ערכנו פ"א על ט' שהוא השלישי והנה תשכ"ט והוא השביעי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ערוך השביעי על התשיעי ויעלה בידך[700] המספר החמש עשרה והוא ד' אלפי אלפים וז' מאות אלף ושמנים ושנים אלף ותתקס"ט[701] והוא החמשה עשר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והקש על זה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והיה זה כן לפי שיחס[702] הראשון אל השני כיחס השני אל השלישי ולזה יהיה שטח הראשון בשלישי כמו שטח השני בעצמו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אבל שטח הראשון בשלישי הוא השלישי לפי שהראשון הוא אחד אם כן שטח השני בעצמו הוא השלישי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ובזה יתבאר ששטח הראשון בחמישי כמו שטח השלישי בעצמו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן יתבאר [703]מה שימשך לזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וגם כן הנה יחס הראשון אל השלישי כמו יחס החמישי אל השביעי אם כן שטח השלישי בחמישי הוא כמו שטח הראשון בשביעי שהוא כמו השביעי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וגם כן הנה יחס הראשון אל השביעי כיחס התשיעי אל החמשה עשר אם כן שטח השביעי בתשיעי הוא כמו שטח הראשון בחמשה עשר שהוא כמו החמשה עשר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והקש על זה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם רצית לדעת מה יעלה המספר האחרון ממספר[704] מונח ממספרים[705] מתיחסים על יחס מונח בלתי מתחילים מן האחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דע תחלה מה יעלה המספר האחרון מהמספר המונח ממספרים מתיחסים על היחס המונח מתחילים מן האחד וערכהו[706] על המספר הראשון וככה המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה שיהיו המספרים המתיחסים חמשה והיחס שלשה והראשון חמשה ורצית לדעת כמה האחרון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה כבר ידעת שהחמישי מזה היחס אם היו מתחילים מן האחד הוא פ"א ערכת פ"א על המספר הראשון שהוא חמשה ועלה ת"ה וככה המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והיה זה כן כי ביחס השווי יחס הראשון שהוא אחד אל[707] החמישי לו כיחס הראשון שהוא חמשה אל החמישי לו מזה היחס | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר המירונו הנה יחס הראשון אל הראשון כיחס החמישי[708] אל החמישי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואולם יחס הראשון אל הראשון הוא חמשה הנה יחס החמישי אל החמישי הוא חמשה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והקש על זה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם רצית לחבר מספר מה ממספרים מתיחסים על יחס מונח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
גרע הראשון מהשני וראה יחס הנשאר מהשני אל הראשון וככה יחס הנשאר מהאחרון כשנגרע ממנו הראשון אל כל המספרים שלפניו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה כבר התבאר בסוף המאמר התשיעי מאקלידס | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה אם רצית לחבר ששה מספרים מתיחסים על יחס ג' והראשון ד' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כבר ידעת שהשני הוא י"ב והאחרון הוא תתקע"ב גרענו מהשני הראשון שהוא ארבעה ונשארו שמנה והנה יחס ד' אל ח' הוא חצי גרענו מהאחרון ד' ונשארו תתקס"ח לקחנו חציים [709]והנה תפ"ד חברנוהו עם תתקע"ב והנה אלף תנ"ו והוא המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Chapter Four – On the Number of Collections of Given Items that Either Differ by the Items or by their Order or by both |
השער הרביעי בחבור מספר מחברות מנושאים מונחים תתחלפנה המחברות בנושאיהם או בסדרן או בשני הענינים יחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If you want to know the number of the collections of a given number of different items that differ only by the order [= permutations]: | אם רצית לדעת מספר מחברות מספר מונח מנושאים מתחלפים המתחלפות בסדר לבד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Take the number that is composed of the successive numbers from one to the given number, and this is the required. | קח המספר המורכב מהמספרים הנמשכים מן האחד עד המספר ההוא וככה המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה אם רצית לדעת בכמה דרכים יתחברו ה'[710] נושאים ותהיינה המחברות מתחלפות בסדר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה המספרים הנמשכים מן האחד עד ה' הם א'ב' ג'ד' ה' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והמספר המורכב ממספרי א'ב' ג'ד' ה' הוא ק"כ וככה המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והיה זה כן לפי שמחברות ב' הם[711] ב' וזה שוה למורכב א'ב' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומחברות שלשה הם כמו שטח ג' בב' שהוא מורכב א'ב'ג' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Likewise it is proven to infinity. | וכזה התבאר זה לאין תכלית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If you want to know the number of collections of a second given number [of items taken out of a set of] a first given number of different items, that differ either by the order or by the items [= variations]: | אם רצית לידע כמה תהיינה מחברות מספר מונח שני ממספר מונח ראשון מנושאים מתחלפים המתחלפים אם בסדר אם בנושאיהן | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| You already know that the [number of] variations of two [of the items] is as the product of the first given number by its preceding number. | כבר ידעת שמחברות השנים הם כמו שטח המספר המונח הראשון במספר הנמשך לו לפניו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומחברות השלשה ממנו יחסם אל מחברות השנים כמו[712] הנשאר מהמספר המונח הראשון כשגרענו ממנו שנים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומחברת הארבעה ממנו יחסם אל מחברות השלשה כמו הנשאר מהמספר המונח הראשון כשגרענו ממנו שלשה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| And so on endlessly. | וכזה ימשך הענין לאין תכלית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Therefore, the procedure is that you take the number that is composed of as many consecutive numbers as the second given number, so that the last one of them should be equal to the first given number; the result is the required. | ומפני זה יהיה הדרך בזה שתקח המספר המורכב מהמספר המונח השני ממספרים נמשכים שיהיה האחרון מהם שוה אל המספר המונח הראשון והעולה הוא המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
[713]דמיון זה אם רצית לדעת מחברות החמשה מח' נושאים מתחלפים אם בסדר ואם בנושאיהן | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה מפני שהמספר המונח השני הוא חמשה תקח מורכב חמשה ממספרים נמשכים שיהיה האחרון מהן שמנה והם[714] ד'ה'ו' ז'ח' והמספר המורכב מהם הוא ו' אלפים תש"כ וככה מחברות החמשה המתחלפות אם בסדר ואם בנושאיהן משמנה נושאים מתחלפים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והיה זה כן לפי שמחברות השנים ממנו הוא שטח ז' בח' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומחברות השלשה ממנו הוא שטח ו' בשטח ז' בח' לפי מה שהתבאר במה שקדם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומחברות הארבעה ממנו הוא שטח ה' במורכב מספרי ו'ז'ח' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומחברות החמשה ממנו הוא שטח ד' במורכב ממספרי ה'ו' ז'ח' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| And so on it is explained endlessly and this is all clear from the above. | וכזה יתבאר לאין קץ וזה כלו מבואר ממה שקדם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If you want to know how many collections there are of a second given number [of items taken out of a set of] a first given number of different items, that differ by the items [= combinations]: | אם רצית לדעת כמה תהיינה מחברות מספר מונח שני המתחלפות בנושאיהן ממספר מונח ראשון מנושאים מתחלפות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Take the number of collections of the second given number [of items taken out of a set of] as many different elements as the first number, that differ either by the order or by the items [= variations] and keep the result. | קח מחברות המספר המונח השני המתחלפות אם בסדר ואם בנושאיהן מהמספר המונח הראשון מנושאים מתחלפים ושמור העולה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Take the number of collections of the second given number [of items] that differ only by the order [= permutation]. | גם קח מחברות המספר המונח השני המתחלפות בסדר לבד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| As the number of times that the reserved counts the result so is the sought. | וכמו שעור אחדי המספר שימנה השמור העולה וככה המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה אם רצית לדעת מחברות החמשה מתחלפות בנושאיהן משמנה נושאים מתחלפים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ראה כמה פעמים ימנה מורכב ד'ה'ו' ז'ח' מורכב א'ב' ג'ד'ה' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה מורכב ד'ה'ו' ז'ח' הוא ו' אלפים תש"כ | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומורכב א'ב' ג'ד'ה' הוא ק"כ | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וו' אלפים תש"כ ימנהו ק"כ נ"ו[715] פעמים והנה נ"ו הוא המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The calculation procedure of this division will be presented afterwards. | וכבר יבא לך דרך חשבון בזאת החלוקה במה שאחר זה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולהקל מעליך כבר ידעת שמחברות החמשה המתחלפות בנושאיהן משמנה נושאים מתחלפים הם כמספר מחברות השלשה המתחלפות בנושאיהן מאלו הנושאים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
[716]ולזה תעיין כמה פעמים ימנה מורכב ו'ז'ח' שהוא של"ו[717] מורכב א'ב'ג' שהוא ו' והנה ימנהו נ"ו פעמים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וככה מחברות השלשה המתחלפות בנושאיהן משמנה נושאים גם ככה מחברות החמשה שהוא שאריתם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The reason for this has already been proven from our saying above. | והסבה בזה כבר התבארה מדברינו במה שקדם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Chapter Five – Division |
השער החמישי בחלק מספר על מספר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| You already know that every product is counted by one of its factors as the number of units of its other factor. | כבר ידעת שכל שטח ימנהו אחד מצלעיו כמספר אחדי הצלע השנית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Therefore, if you know the number of the product and you know one of its factors, you can calculate the other factor. | על כן אם ידעת מספר השטח וידעת אחת מצלעותיו תוכל להוציא הצלע השנית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The procedure for this is that you write the number of the product in one row and beneath it in another row you write the known factor, then divide the top row by the bottom row, and the result is the second factor. | והנה אופן המעשה בזה שתכתוב מספר השטח בטור אחד ותחתיו תכתוב בטור אחר הצלע הידוע ותחלוק הטור העליון על הטור השפל והעולה בידך הוא הצלע השנית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| But how you should divide the top row by the bottom row? This is as I will tell you: | ואולם איך תחלוק הטור העליון על הטור השפל הנה כפי מה שאומ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| First, look at the last digit in the bottom row and the digit in the preceding rank, and consider all the digits that precede the preceding rank of the bottom row as if they were one alone in the preceding rank. | תסתכל תחלה במספר האחרון שבטור התחתון ובמספר שבמעלה הקודמת לו וכל המספרים שלפני המעלה הקודמת לו בטור התחתון תחשוב כאלו הם אחד לבד במעלה הקודמת לו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The units you have in the last rank of the bottom row are considered as units. | ומה שיעלה בידך מן האחדים במעלה האחרונה שבטור התחתון יהיו לאחדים בידך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The units you have in rank that precedes the last rank are considered as tenths of one integer. | ומה שיעלה בידך מן האחדים במעלה שלפני האחרונה יהיו לעשיריות אחד שלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Keep what the units and the tenths that you have. | ומה שיהיה בידך מן האחדים והעשיריות שמרם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Then, look at the last digit in the top row, it will be as units for you. | אח"כ התבונן במספר התחתון שבטור העליון והיו לאחדים בידך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The units that are in the preceding rank will be as tenths. | והאחדים שבמעלה שלפניו יהיו עשיריות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Do not consider the other digits. | ולא תחוש לשאר המספרים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If the units and the tenths that you have received are more than the reserved or as much as the reserved, calculate how many times the reserved is contained in it in its entirety, then put the result in a middle row between the two rows in the rank, whose distance from the last rank of the upper row is as the distance of the last rank of the bottom row from the rank of units. | והעולה בידך מן האחדים והעשיריות אם הם יותר מהשמור או כדי השמור תחשוב כמה פעמים יהיה בו השמור בשלמות והעולה בידך תשימהו בטור אמצעי בין שני הטורים במעלה אשר מרחקה מהמעלה האחרונה שבטור העליון לפניו כמרחק המעלה האחרונה שבטור התחתון ממדרגת האחדים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Then multiply that digit that is in the result row by the bottom row, subtract the product you get from the top row, write the remainder over the top row and erase the previous [digit in the] top row. | אחר כך ערוך המספר ההוא אשר בטור העולה על הטור התחתון והעולה בידך תגרענו מהטור העליון ותכתוב הנשאר בידך על הטור העליון ותמחוק הטור העליון הקודם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If the last digit of the top row with the tenths that were there are not as much as the reserved, lower the last rank to the preceding one, consider the units and the tenths of the preceding and see how many times the result is counted by the reserved in its entirety, write the result in the rank, whose distance from the last rank of the top row after the lowering is as the distance of the last rank of the bottom row from the first rank, then proceed as the previous procedure. | ואם לא היה המספר האחרון אשר בטור העליון עם העשיריות אשר שם כמו השמור תוריד המעלה האחרונה אל שלפניה וממנה תחשוב האחדים והעשיריות משלפניה והעולה בידך תראה כמה פעמים ימנהו השמור בשלמות והעולה תכתוב במעלה אשר מרחקה לאחור מהמעלה האחרונה אחר ההורדה שבטור העליון כמרחק המעלה האחרונה שבטור התחתון מהמדרגה הראשונה ותנהג הענין על המנהג הקודם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Then, do with what is left of the top row as you did with the previous top row. | אח"כ תשוב לעשות מהטור העליון הנשאר בידך כמו מה שעשית מהטור העליון הקודם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Proceed like this until nothing is left in the top row, or until you are left with less than the bottom row if the top row does not count the bottom row. | וכן תעשה עד שלא ישאר לך בטור העליון דבר או שישאר לך פחות מהטור התחתון אם היה שלא ימנה הטור העליון הטור התחתון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| In the following we will tell you what to do with this remainder. | ועוד נודיעך במה שיבא מה תעשה מהנשאר ההוא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Sometimes it happens that we have to write the result twice in one rank, but it is rare that it happens. | ופעמים יקרה שנצטרך לכתוב בטור העולה שתי פעמים במעלה אחת ומעט מה שיקרה זה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה רצינו לחלק טור א'ב'ג'ד'ה'ו'ז'ח'ט' על טור ז'ג'ד'ט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה המספר האחרון שבטור התחתון והוא ז'ג'ד'ט' הוא ט' והם שלמים ובמעלה שלפניו מספר ד' וכל מה שלפניו הוא אחד בה על צד האומד והקרוב ויהיו ה' והם עשיריות ולזה יהיה השמור ט' שלמים וה' עשיריות והנה ט' שלמים וח' עשיריות ימנם ט' שלמים וה' עשיריות פעם אחת ולזה נכתוב א' בטור האמצעי בין שני אלו הטורים במעלה הרביעית לאחרונה שבטור העליון לפניה לפי שמספר האחרון שבטור התחתון הוא מרביעית ולפי זה יהיה הא' שבטור האמצעי מהמעלה הששית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הכינו א' על הטור התחתון ועלה ז' מהששית ג' מהשביעית ד' מהשמינית ט' מהתשיעית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
גרענו העולה מהטור העליון ונשאר בטור העליון א'ב'ג'ד'ה'ט'ג'ד' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונכתוב זו על הטור העליון ונמחק הטור הקודם והנה במעלה האחרונה שבטור העליון עם מה שלפניו ד' שלמים וג' עשיריות והוא פחות מהשמור ולזה תוריד ד' אל שלפניו יהיו מ' בה וג' שהיו בה והנה מ"ג וט' עשיריות במעלה שלפניו חלקנו מ"ג וט' עשיריות על ט' וה' עשיריות שהוא השמור ועלה ד' בשלמות ונכתוב ד' בטור האמצעי במעלה הרביעית לאחור למעלת המ"ג ולזה יהיו הד' מהרביעית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הכינו ד' על הטור התחתון ועלה ח' ברביעית ד' בחמשית ז' בששית ז' בשביעית ג' בשמינית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
גרענו העולה מהטור העליון השני ונשאר א'ב'ג'ו'0ב'ו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה מה שבמעלה האחרונה עם מה שבמעלה שלפניה הוא פחות מהשמור ולזה נוריד הו' שבמעלה האחרונה אל שלפניה יהיו ששים בה וב' שהיו בה והנה ס"ב ואין במעלה שלפניה דבר נחלק ס"ב על השמור ועלה ו' ונשימם בשלישית לפי שהיו ברביעית לאחור ממעלת ס"ב שחלקנו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ערכנו ו' מהשלישית על הטור התחתון ועלה ב' מהשלישית ב' מהרביעית ו' בחמשית ו' בששית ה' בשביעית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
גרענו העולה מהטור העליון השלישי ונשאר הטור העליון הרביעי א'ב'א'ד'ד'ה' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונוריד ה' אל המעלה שלפניה וד' אשר בה והנה נ"ד וד' שבמעלה שלפניה יהיו ד' עשיריות חלקנו נ"ד וד' עשיריות על השמור ועלה ה' ונשימם בטור האמצעי בשנית שהיא הרביעית לאחור מהמעלה שחלקנו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ערכנו ה' מהשנית על הטור התחתון ועלה ה'ח'א'ז'ד' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
גרענו העולה מהטור העליון הרביעי ונשאר א'ז'ב'ב'ז' והוא הטור העליון החמשי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונוריד ז' אל המעלה שלפניה וב' אשר בה והנה ע"ב חלקנו על השמור ועלה ז' ונשימם בטור האמצעי בראשונה שהיא הרביעית לאחור מהעלה אשר חלקנו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ערכנו ז' מהראשונה על הטור התחתון ועלה ט'ה'0ו'ו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
גרענום מהטור העליון החמשי ונשאר ב'א'ב'ו' והוא פחות מהטור התחתון והוא הנשאר שלא הגיע לחלוק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה העולה בטור האמצעי הוא ז'ה'ו'ד'0א' והנשאר בטור העליון הוא ב'א'ב'ו' וכתבנום בטור ששי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והקש על זה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| You should know that if the numbers in the upper row, up to the rank whose distance from the last rank of [that row] is the same as the distance of the last rank of the lower row from the first, when we lower all of them down to that rank, are the same as the numbers of the lower row, when we lower them down to the first rank, or greater, then you should write 1 in the row of the result, in the appropriate rank according to what preceded, even if you do not get in the upper row [the exact number] in the way we indicated. | וראוי שתדע שאם היו המספרים אשר בטור העליון עד המעלה אשר מרחקה מהמעלה האחרונה ממנו כמרחק המעלה האחרונה שבטור התחתון מן הראשונה כשהורדנום כלם אל המעלה ההיא כמספר הטור התחתון כשהורדנוהו אל המעלה הראשונה או יותר הנה ראוי שתשים אחד בטור העולה במעלה הראויה לפי מה שקדם ואע"פ שלא תשיג בטור העליון על הצד שהיישרנו בשלמות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
משל שיהיה לך לחלק טור ג'ז'ד'ט' על טור ג'ד'ט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ויהיה השמור לפי מה שהיישרנו אליו ט' אחדים וה' עשיריות ובטור העליון ט' אחדים וד' עשיריות ולזה יחשב לפי מה שקדם שיצטרך להוריד ט' אל שלפניו ואמנם כשהורדנו ג'ד'ט' שבטור העליון אל המעלה השלישית לאחור למעלת ט' היו תשע מאות ומ"ג וכזה מספר הטור התחתון כשהורד אל הראשונה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולזה תשים בטור העולה אחד במעלה השניה ותערכהו על הטור התחתון ותגרע העולה מהטור העליון ולא ישאר לך בטור העליון כי אם ז' מהראשונה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והקש על זה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| You should apply this, when the last number in the bottom row is equal to the last number in the upper row and the number that precedes it is equal to the number that precedes the other [in the upper row]. | ואתה צריך לחוש לזה כשיהיה המספר האחרון שבטור התחתון שוה למספר האחרון שבטור העליון והמספר השני לו לאחור שוה למספר השני אל האחור לאחר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Since we should explain what should be done with the remainder in the upper row, and there is no way to explain it, without first explaining the way to multiply fractions by integers, let us first explain all the ways of multiplying fractions and fractions of fractions. | ולפי שראוי שנבאר מה יעשה מהנשאר בטור העליון ואין דרך לזה הביאור אלא אם כן התבאר קודם דרך כפל השברים בשלמים הנה נבאר ראשונה דרך כפל השברים ושברי שברים בכל אופניהם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Fractions |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Simple Fractions |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Know that the ratio of each fraction to one is as the ratio of one to the denominator of that fraction. | דע שיחס כל חלק אל אחד כיחס אחד אל המספר המורה אל החלק ההוא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והמשל שיהיה יחס שלישית אל אחד כיחס אחד אל שלשה שהוא מורה על שלישית לפי שהאחד הוא שלישית שלשה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| When this is established it is proven that the ratio of the product of a given fraction by one to the product of one by one is as the ratio of one to the denominator of the given fraction. | וכאשר התישב זה הנה יתבאר שיחס שטח שבר מונח באחד אל שטח אחד באחד הוא כיחס אחד אל המורה על השבר המונח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והמשל שיחס שטח שלישית באחד אל שטח אחד באחד הוא כיחס אחד אל שלשה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה שיחס שטח שלישית באחד אל שטח אחד באחד הוא כיחס שלישית אל אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אבל יחס שלישית אל אחד הוא כיחס אחד אל שלשה ויחס אחד אל שלשה הוא שלישית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
א"כ שטח שלישית באחד הוא שלישית אחד לפי ששטח אחד באחד הוא אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| It is also proven by a similar explanation that the product of a given number of integers or one integer by a given number of fractions or one fraction, is as many parts of the denominator as the product of the multiplier by the multiplicand. | וגם כן יתבאר בכמו זה הביאור ששטח מספר מונח משלמים או שלם אחד במספר מונח משברים או בשבר אחד הם חלקים מהמורה באחד כמספר שטח מספר המכה במספר המוכה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והמשל שנרצה להכות ה' שביעיות על מ' שלמים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונאמר שהעולה הוא חלקים משבע באחד כמספר שטח ה' במ' שהוא ר' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והוא שיחס שטח ה' שביעיות במ' שלמים אל שטח שביעית באחד מחובר משני יחסים מיחס חמשה אל אחד ומיחס מ' אל אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה היחס המחובר משני אלו היחסים הוא יחס מאתים אל אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אבל שטח שביעית באחד הוא שביעית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם כן שטח ה' שביעיות במ' שלמים הוא ר' חלקים משבעה באחד שהם כ"ח שלמים וד' שביעיות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| There is no doubt that by this same explanation the matter is proven if there is a number only in one of them, i.e. in the fractions or in the integers. | ואין ספק שבזה הביאור בעצמו התבאר הענין אם לא היה מספר כי אם באחד מהם ר"ל בשברים או בשלמים והקש על זה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| After the method of multiplication of fractions by integers has been explained in all ways, we shall explain the method of multiplication of fractions by fractions. | ואחר שהתבאר אופן הכאת שברים בשלמים בכל אופניהם הנה נבאר אופן הכאת שברים בשברים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| We shall explain that the ratio of the product of a fraction by a fraction to one is as the ratio of one to the product of one denominator by the other denominator. | ונבאר שיחס שטח שבר בשבר אל אחד כיחס אחד אל השטח ההוה מהמורה האחד במורה האחר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והמשל שיחס שטח שלישית בחמשית אל אחד כיחס אחד אל שטח שלשה בחמשה שהוא ט"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה שיחס שטח שלישית בחמשית אל שטח אחד באחד שהוא אחד מחובר משני יחסים מיחס שלישית אל אחד ומיחס חמשית אל אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן יחס שטח אחד באחד שהוא אחד אל שטח שלשה בחמשה מחובר משני יחסים מיחס שלישית אל אחד ומיחס חמשית אל אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכבר היה יחס שטח שלישית בחמשית אל שטח אחד באחד מחובר משני אלו היחסים בעצמם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
א"כ יחס שטח שלישית בחמשית אל אחד כיחס אחד אל שטח שלשה בחמשה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אבל יחס אחד אל שטח שלשה בחמשה הוא חלק מט"ו באחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
א"כ שטח שלישית בחמשית הוא חלק מט"ו באחד והקש על זה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ובזה התבאר ששטח שלישית בשלישית הוא תשיעית אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Thus, it is proven by a similar explanation that the product of a given number of fractions or one fraction by a given number of fractions or one fraction, is as many parts of the product of one denominator by the other as the product of the multiplier by the multiplicand. | ובזה יתבאר בכמו זה הביאור ששטח מספר מונח משברים מה או שבר מה במספר מונח משברים מה או בשבר מה הוא חלקים משטח המורה האחד באחר כמספר שטח המספר המכה במספר המוכה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והמשל שנרצה להכות ד' שביעיות על ה' תשיעיות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונאמר שהעולה הוא חלקים מס"ג באחד שהוא שטח המורה האחד באחר כמספר שטח ד' בה' שהוא כ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה שיחס שטח שביעית בתשיעית אל שטח ד' שביעיות בה' תשיעיות מחובר משני יחסים מיחס אחד אל ד' ומיחס אחד אל ה' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אבל זה היחס המחובר הוא יחס אחד אל כ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם כן יחס שטח שביעית בתשיעית אל שטח ד' שביעיות בה' תשיעיות הוא יחס אחד אל כ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אבל שטח שביעית בתשיעית הוא חלק מס"ג חלקים באחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
א"כ ד' שביעיות בה' תשיעיות הוא כ' חלקים מס"ג חלקים באחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| והקש על זה | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| There is no doubt that by this same explanation it is proven if there is a number only in one of them. | ואין ספק שבזה הביאור בעצמו יתבאר אם לא היה מספר כי אם באחד מהם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| When all of this is clear, we shall tell you a way to find the denominator of a fraction of a fraction. | וכאשר התבאר זה כלו נודיעך דרך לקיחת המורה בשבר השבר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| We say that the denominator of a fraction of a fraction is the number that consists of the denominators of these fractions. | ונאמר שהמורה על שבר השבר הוא המספר המורכב מהמספרים המורים על השברים ההם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והמשל ששלישית חמשית שלישית הוא אחד ממורכב ממספרי שלשה חמשה שלשה באחד ולזה יהיה חלק ממ"ה באחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה שיחס שטח חמשית בשלישית אל שטח אחד באחד כיחס שטח אחד באחד אל שטח חמשה בשלשה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם כן חמשית שלישית הוא חלק מט"ו באחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וגם כן הנה יחס שטח שלישית בחלק מט"ו באחד אל שטח אחד באחד כיחס שטח אחד באחד אל שטח שלשה בט"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
א"כ שלישית חמשית שלישית הוא חלק ממ"ה באחד והקש על זה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| And after we know the denominator, you can apply the multiplication in the previous way for both integers and fractions. | ואחר שידענו המורה תנהיג ההכאה באופן הקודם בין בשברים בין בשלמים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה אם רצינו להכות ה' שביעיות שלישיות על ז' שמיניות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה המורה לשביעית שלישית הוא כ"א והמורה לשמינית הוא שמנה והמורכב משני המורים הוא קס"ח ערכנו ה' על ז' ועלה ל"ה והם ל"ה חלקים מקס"ח באחד שלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| והקש על זה | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The way of addition of various fractions: | דרך חבור השברים השונים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Take the smallest number that is counted by [= common multiple] all the denominators of these fractions and this is the denominator. Take from it these fractions in their entirety, and you divide the result by the denominator; this is the sought for. | קח המספר המעט שימנוהו כל המורים לשברים ההם והוא המורה הנה וממנו תקח השברים ההם בכללם והעולה תחלקהו על המורה והוא המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה אם רצינו לחבר ב' שלישיות עם ד' חמשיות ועם ה' ששיות ועם ג' רביעיות ששית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כבר ידענו שהמספר המעט שימנוהו שלשה וה' וו' וכ"ד הוא ק"כ וכבר התבאר דרך לקיחתו מאקלידס | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה ב' שלישיותיו הם פ' וזה יתבאר כשנחלק ק"כ על שלשה ונקח ב' דמיוני העולה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וד' חמשיותיו הם צ"ו וה' ששיותיו הם ק' וג' רביעיות ששיותיו הם ט"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חברנו כל אלו המספרים ועלה רצ"א חלקנו רצ"א על ק"כ ועלה ב' שלמים נ"א חלקים מק"כ באחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והיה זה כן לפי שיחס ב' שלישיות אחד אל אחד כיחס ב' שלישיות ק"כ אל ק"כ | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכזה יתבאר בנשאר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר קבצנו הנה יחס כל אלו השברים אל אחד כיחס רצ"א אל ק"כ | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| והקש על זה | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The way to know the result of multiplication of fractions by fractions or by fractions of fractions: | דרך לדעת העולה מהכאת השברים בשברים או בשברי השברים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| You already know that the product of fractions by fractions are parts of the denominator that is composed of their denominators, and it has already been proven that the number that is composed of some numbers is counted by one of the numbers as many times as the units of the number that is composed of the other numbers, and it is also counted by the number that is composed of some of its numbers as many times as the units of the number that is composed of the remaining numbers. | כבר ידעת שהכאת השברים בשברים הם חלקים מהמורה המורכב ממוריהם וכבר התבאר שהמספר המורכב ממספרים מה ימנהו אחד מהמספרים בכמו אחדי המספר המורכב מהמספרים הנשארים וכן ימנהו המספר המורכב ממספרים מה ממנו בכמו אחדי המספר המורכב מהמספרים הנשארים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Therefore, if you divide the number you have by one of the numbers, the result will be parts of the number that is composed of the remaining [numbers]. | ובהיות הענין כן אם תחלק המספר אשר בידך על אחד מהמספרים יהיה העולה חלקים מהמספר המורכב מהנשארים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
משל זה אם תכה ו' שביעיות על ז' שמיניות עלה מ"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תחלק מ"ב על שבעה יהיה העולה בידך שמיניות לפי שהמספר המורכב מז' וח' ימנהו ז' כשיעור אחדי ח' ולזה יהיה העולה ו' שמיניות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תחלק על שמנה יעלה בידך שביעיות ולזה יהיה העולה ה' שביעיות וב' שמיניות שביעית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון אחר אם רצית להכות כ"ח חלקים מכ"ט באחד על ו' שביעיות שלישית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תערוך כ"ח על ו' ועלה קס"ח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה אם תחלק קס"ח על כ"ט יעלו בידך שביעיות שלישית שהם השברים הנשארים ויהיה העולה ה' שביעיות שלישית וכ"ג חלקים מכ"ט שביעיות שלישית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם חלקת על המספר המורכב מז' וג' יעלו בידך חלקים מכ"ט ויהיה העולה ח' חלקים מכ"ט באחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תחלוק על ז' יעלו בידך שלישיות חלק מכ"ט חלקים באחד ולזה יהיה העולה כ"ד שלישיות חלק מכ"ט באחד ולזה יהיה העולה ח' חלקים מכ"ט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תחלוק על ג' יעלו בידך שביעיות חלק מכ"ט חלקים באחד ולזה יהיה העולה נ"ו שביעיות חלק מכ"ט חלק באחד שהם ח' חלקים מכ"ט באחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| והקש על זה | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The way to multiply fractions by fractions and the result by fractions and so on: | דרך להכות שברים על שברים והעולה על שברים וכן מה שיהיה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Multiply the number of the first fractions by the number of the second fractions, then multiply the result by the number of the third fractions and so on until all fractions are complete. | ערוך מספרי השברים הראשונים על מספרי השברים השניים והעולה בידך ערכהו על מספר השברים השלשיים וכן עד כלות כל השברים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Then, divide the result by the denominator of one of the fractions or by the product of the denominators of some of them, the result that you get are some of parts of the product of the denominators of the remaining fractions - you choose the most convenient by which to divide. | אחר כך תחלוק העולה על המורה לאחד מהשברים או על המורכב ממספרי מורים מה מהם והעולה בידך יהיו חלקים מה ממורכב מהמורים לשברים הנשארים ואתה תבחר היותר נכון לחלק עליו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה נרצה להכות ו' שביעיות על ה' ששיות והעולה על ג' רביעיות והעולה על ז' שמיניות והעולה על ב' שלישיות והעולה על ב' שביעיות והעולה על שלישיות שלישית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הכינו ו' על ה' ועלה ל' הכינו ל' על ג' ועלה צ' הכינו צ' על ז' ועלה תר"ל הכינו תר"ל על ב' ועלה אלף ור"ס הכינו אלף ור"ס על ב' ועלה אלפים ותק"כ ערכנו אלפים ותק"כ על אחד ועלה אלפים ותק"כ | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה השברים אשר בידך הם שביעית ששית רביעית שמינית שלישית שביעית שלישית שלישית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תחלק אלפים ותק"כ על המורה לאחד מאלו השברים יהיה העולה בידך חלקים מהמספר המורכב ממורי השברים הנשארים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה מפני שהמספר רב ראוי שתחלקהו על המספר המורכב מקצת המורים אשר יראה בעיניך שהוא יותר נאות לחלק עליו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקנו אותו על המורכב משבעה וו' וג' וד' שהוא תק"ד ועלה ה' והם חלקים ממורכב מורי השברים הנשארים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואולם הנשארים הם שמינית שביעית שלישית שלישית א"כ העולה מזאת ההכאה הם ה' שמיניות שביעית שלישית שלישית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| והקש על זה | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והיה זה כן לפי שכבר יתבאר ממה שקדם במעט עיון שהעולה שהוא אלפים תק"כ הם חלקים ממורכב מכל מורי השברים באחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Understand and you will find it so. | והבן ותמצא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Another easier way for this: know that the number that is composed of given numbers and given fractions, its ratio to one is the ratio that is composed of the ratios of the numbers of these fractions when the are set as antecedents to the numbers of their denominators that are set later. | דרך אחרת קלה לזה דע כי המספר המורכב ממספרים מונחים ומשברים מונחים יחסו אל אחד היחס המחובר מיחסי מספרי השברים ההם כשיושמו קודמים אל מספרי מוריהם כשיושמו נמשכים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
משל זה שיחס הכאת שלשה שביעיות בד' חמשיות והעולה בב' חמשיות רביעית להכאת אחד באחד והעולה באחד שהוא אחד לעולם כמה שהגיע הכפל הוא מחובר משלשה יחסים מיחס שלשה שביעיות אל שבעה שביעיות שהוא אחד ומיחס ארבעה חמשיות אל ה' חמשיות שהוא אחד ג"כ ומיחס ב' חלקים מי"ב אל י"ב חלקים מי"ב שהוא אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| You already know that when the order of the former or the last or both is switched, the composite ratio remains the same. | וכבר ידעת שכאשר הומר סדור הקודמים או הנמשכים או שניהם יחד נשאר היחס המחובר בעינו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If so, when you have to multiply a number of fractions by a number of fractions and so on, you can switch the numbers of these fractions with another number of the other fractions, if it is more convenient for the multiplication. | ובהיות הענין כן אם היה לך להכות מספר שברים על מספר מה משברים וכן מה שהגיע ההרכבה תוכל להמיר מספרי אלה השברים במספר אחר מהשברים האחרים אם היה יותר נאות אל ההכאה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
משל זה שאם היה לך להכות ג' שביעיות על ז' שמיניות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תוכל להמיר השבעה אל השביעיות ויהיה לך להכות ג' שמיניות על ז' שביעיות שהוא אחד והעולה הוא ג' שמיניות וככה המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון אחר אם רצית להכות ד' שביעיות על ה' שמיניות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תוכל להמיר הד' אל השמיניות ויהיה לך להכות ד' שמיניות שהוא חצי אחד על ה' שביעיות ויעלה ב' שביעיות וחצי וככה המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון אחר אם היה לך להכות ג' רביעיות על ד' חמשיות והעולה על ו' שביעיות והעולה על ז' שמיניות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תמיר המספרים שהם ג' ד' ו' ז' במקומות היותר נאותים ולזה תשים הז' אצל השביעיות והד' אצל הרביעיות והו' אצל השמיניות והג' אצל החמשיות ולזה תכה ז' שביעיות שהוא אחד על ד' רביעיות שהוא אחד ויעלה אחד והעולה שהוא אחד תכה על ו' שמיניות ויהיו בידך ג' רביעיות והעולה תכה על ג' חמשיות ויעלה בידך ט' רביעיות חמשית ואם תחלקנו על ארבעה יהיה העולה חמשיות ויעלה בידך ב' חמשיות ורביעית חמשית ואם תחלקנו על ה' יהיה העולה רביעיות ויעלה בידך רביעית וד' חמשיות רביעית וככה העולה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון אחר אם היה לך להכות ג' חמשיות על ששית והעולה על ז' שמיניות והעולה על ד' תשיעיות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תמיר המספרים שהם ג' ד' ז' במקומות היותר נאותים ולזה תשים הג' אצל הששיות וארבעה אצל השמיניות והז' אצל התשיעיות וישאר חמשית בזולת מספר ותכה ג' ששיות שהם חצי על ארבעה שמיניות שהם חצי ויעלה בידך רביעית תכהו על חמשית ויהיה רביעית חמשית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| והקש על זה | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The way to multiply some fractions by various fractions: | דרך הכאת שברים מה בשברים שונים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If you have to multiply a given fraction or a number of given fractions by given fractions, multiply the given fraction or the given fractions by the first type of the various fractions, divide the result by the denominator of the multiplicand fraction, so that the result is parts of the multiplier. Do so until the first multiplier is multiplied by all the successive the [multiplicands], then all parts you have in the result are fractions of the multiplier. | אם היה לך להכות שבר מונח או מספר שברים מונחים על שברים מונחים ערוך השבר המונח או השברים המונחים על המין הראשון מהשברים השונים וחלק העולה על המורה על השבר המוכה כדי שיהיה העולה בידך חלקים מהמכה וכן תעשה עד שיוכה הראשון המכה על כל הנמשכים ובזה יהיו כל החלקים אשר בידך בעולה משברי המכה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון רצינו להכות ב' שלישיות על ו' שביעיות ועל ז' שמיניות ועל ח' תשיעיות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נכה ב' על ו' יהיו י"ב נחלקם על שבעה שהוא מורה לשבר המוכה ועלה אחד וה' שביעיות והוא שלישית וה' שביעיות שלישית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ערכנו ב' על ז' ועלה י"ד נחלקם על שמנה שהוא שבר המוכה ועלה א' וו' שמיניות והוא שלישית וג' רביעיות שלישית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ערכנו ב' על ח' ועלה ט"ז נחלקם על תשעה ועלה אחד וז' תשיעיות והוא שלישית וז' תשיעיות שלישית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חברנו כל השברים אשר בעולה שהם ג' שלישיות וה' שביעיות שלישית וג' רביעיות שלישית וז' תשיעיות שלישית ועלה אחד שלם וב' שלישיות וס"א חלקים מתשנ"ו באחד והקש על זה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If you have to multiply integers and fractions, as many as they may be, by integers and fractions, as many as they may be, you already know the method of multiplication of integers by integers as well as the method of multiplication of integers by fractions and the method of multiplication of fractions by fractions. Therefore, you multiply all the integers of the multiplier row by all of the integers and the fractions of the multiplicand row; then, multiply all the fractions of the multiplier row by all the integers and the fractions of the multiplicand row. | ואם היה לך להכות שלמים ושברים כמה שיהיו על שלמים ושברים כמה שיהיו הנה כבר ידעת אופן הכאת שלמים בשלמים ואופן הכאת שלמים בשברים ואופן הכאת שברים בשברים ולזה תכה כל השלמים שבטור המכה על כל השלמים והשברים אשר בטור המוכה עוד תכה כל השברים שבטור המכה על כל השלמים והשברים אשר בטור המוכה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה אם היה לך להכות י"ב וג' חמשיות וד' תשיעיות על כ"א וב' שלישיות וג' רביעיות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הכה י"ב על כ"א ועלה רנ"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הכה י"ב על ב' שלישיות ועלה ח' שלמים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הכה י"ב על ג' רביעיות ועלה ט' שלמים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הכית ג' חמשיות על כ"א שלמים ועלה י"ב שלמים וג' חמשיות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הכית ג' חמשיות על ב' שלישיות ועלה ב' חמשיות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הכית ג' חמשיות על ג' רביעיות ועלה ב' חמשיות ורביעית חמשית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הכית ד' תשיעיות על כ"א ועלה ט' שלמים ושלישית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הכית ד' תשיעיות על ב' שלישיות ועלה ב' תשיעיות וב' שלישיות תשיעית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הכית ד' תשיעיות על ג' רביעיות ועלה שלישית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חברנו כל העולה ועלה רצ"ו שלמים וב' חמשיות וז' חלקים מתק"ם באחד וזה המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| This is the rule if there are fractions of fractions, because you already know the way of their multiplication. | וכן ההקש אם היו שם שברי שברים כי כבר ידעת אופן הכאתם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Sometimes you need a lot of operations, such as when you have to multiply a number of integers and fractions and products of fractions by fractions or by integers also by integers and fractions and products of fractions by fractions. | ופעמים תצטרך למלאכות רבות כמו שיהיה לך להכות מספר שלמים ושברים ושטח שברים בשברים או בשלמים גם כן על שלמים ושברים ושטח שברים בשברים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The way of this is that you first find the multiplier by knowing the result of that product and adding it to the integers and the fractions. In short, you first sum all that is in the multiplier. Then, you sum what is in the multiplicand and multiply the first row by the other according to the previous way. | והדרך בזה שתוציא ראשונה המספר המכה כשתדע העולה מהשטח ההוא ותחברהו עם השלמים והשברים וסוף דבר תחבר ראשונה כל מה שבמכה עוד תחבר מה שבמוכה ותכה אח"כ הטור האחד על האחר באופן הקודם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If you have to subtract fractions, as many as they may be, of various [types of] fractions, take the smallest number that is counted by [= common multiple of] the denominators of all the fractions, and it is the [common ] denominator. Take from it the fractions that you want to subtract and put them in a row. Then, take from it the fractions from which you want to subtract, and subtract the other row from them. What you have left are parts of the denominator that we take. That is clear. | ואם היה לך לגרוע שברים כמה שיהיו משברים שונים מהם תקח המספר המעט שימנוהו המורים לכל השברים והוא המורה הנה וממנו תקח השברים שתרצה לגרוע ותשימם בטור אחד עוד תקח ממנו השברים שתרצה לגרוע מהם ומהם תגרע הטור האחר והנשאר בידך הם חלקים מהמורה אשר לקחנו וזה מבואר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Another way to multiply integers and fractions by integers and fractions: | דרך אחרת לכפול שלמים ושברים על שלמים ושברים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Take the smallest number that is counted by [= common multiple of] the denominators of all the fractions, and it is the [common ] denominator. Multiply it by the multiplier row and set that result in a first row. Multiply the multiplicand row also by the denominator and put the result in a second row. Multiply the top row by the bottom row and the result you get is the sought after. | קח המספר המעט שימנוהו המורים לכל השברים והוא המורה הנה ועליו תערוך הטור המכה ותשים העולה בטור אחד ראשון גם על המורה תכה הטור המוכה ותשים העולה בטור אחד שני ותערוך הטור העליון על הטור השפל והעולה בידך הוא המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והמשל אם היה לך להכות י"ב וג' חמשיות וד' תשיעיות על כ"א וב' שלישיות וג' רביעיות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה ידעת שהמספר הראשון שימנה כל אחד מאלו השברים הוא ק"פ | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
לקחת י"ב דמיוני ק"פ וג' חמשיות ק"פ וד' תשיעיות ועלה ב' אלפים וג' מאות וארבעים ושמנה ותשימם בטור אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
לקחת כ"א דמיוני ק"פ וב' שלישיותיו וג' רביעיותיו ועלה ד' אלפים ול"ה ונשימם בטור האחר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הכית הטור האחד על הטור האחר ועלה 0ח'א'ד'ז'ד'ט' חלקת העולה על מרובע ק"פ ועלה רצ"ב שלמים ונשארו 0ח'ג'ג'א' והם חלקים ממרובע ק"פ באחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר נבחן תמצא הנשאר ב' חמשיות ות"ך חלקים ממרובע ק"פ שהם ב' חמשיות וז' חלקים מתק"ס וזה מסכים לחשבון הראשון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| והקש על זה | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והיה זה כן לפי שנכפל כל אחת מצלעות השטח על ק"פ היה יחס השטח אל השטח יחס צלעו אל צלעו שנוי ביחס לפי שהשטחים מתדמים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אבל יחס צלעו אל צלעו הוא ק"פ | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם כן יחס השטח אל השטח הוא כמו מרובע ק"פ | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם כן השטח אשר צלעיו נכפלים על ק"פ ימנהו מרובע ק"פ במספר אחדי השטח הראשון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| After the way of multiplication of fractions in all their types have been proven, we shall explain you what to do with what cannot be divided and the way is that you divide as much as you can divide, and the remainder are parts, of which the number of the row by which you divide is one. | ואחר שהתבאר אופן כפל השברים בכל מיניהם הנה נבאר לך מה תעשה ממה שלא הגיע לחלוק והדרך בזה שתחלוק כל מה שתוכל לחלקו והנשאר הם חלקים מהמספר הטור שתחלוק עליו באחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה אם רצית לחלק נ"ג על י"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
יעלה ג' וישארו י"א והם י"א חלקים מי"ד באחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והיה זה כן לפי שהחלק מי"ד כשהוכה על י"ד היה י"ד חלקים מי"ד באחד שהוא אחד שלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם כן הי"א חלקים כשהוכו על י"ד היו י"א שלמים והוא מה שנשאר לנו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| והקש על זה | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If you want to divide integers and fractions by integers and fractions, take the common denominator of all the fractions and multiply by it the whole row you want to divide, put the result in a row, then multiply by the [common] denominator the row, by which you want to divide, put the result in a second row under the first row, so that you can put the result row between the two rows, and when this is complete, divide the upper row by the lower row, and the result is the sought after. | ואם רצית לחלק שלמים ושברים על שלמים ושברים קח המורה הראשון למורה כל השברים ועליו תערוך הטור שרצית לחלק ותשים העולה בטור אחד עוד תערוך על המורה הטור שרצית לחלק עליו ותשים העולה בטור שני תחת הטור האחד בדרך שתוכל לשום טור העולה בין שני הטורים וכאשר ישלם זה חלק הטור העליון על הטור התחתון והעולה הוא המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה אם רצית לחלק פ"ד וג' חמשיות וג' רביעיות על י' וב' שלישיות וג' שמיניות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה המורה הראשון לכל אלו השברים הוא ק"כ | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ערכנו פ"ד וג' חמשיות וג' רביעיות על ק"כ ועלה עשרת אלפים רמ"ב והוא הטור שתחלק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ערכנו י' וב' שלישיות וג' שמיניות על ק"כ ועלה אלף שכ"ה והוא הטור שתחלק עליו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקת הטור העליון על הטור התחתון ועלה ז' שלמים ותתקס"ז חלקים מאלף שכ"ה באחד וככה המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והיה זה כן לפי שכבר לוקחו לפ"ד וג' חמשיות וג' רביעיות והוא הראשון ולמספר י' וב' שלישיות וג' שמיניות והוא השני כפלים שוים והם ק"כ | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם כן יחס כפלי הראשון הלקוחים אל כפלי השני כיחס הראשון אל השני | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אבל יחס כפלי הראשון אל כפלי השני הוא ז' שלמים ותתקס"ז חלקים מאלף שכ"ה באחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם כן יחס הראשון אל השני הוא ז' שלמים ותתקס"ז חלקים מאלף שכ"ה באחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| והקש על זה | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תרצה לבחון זה הכה ז' שלמים ותתקס"ז חלקים מאלף שכ"ה באחד על י' וב' שלישיות וג' שמיניות ויצא לך פ"ד וג' חמשיות וג' רביעיות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Sexagesimal Fractions |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Multiplication of sexagesimal fractions |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Having explained this, we should explain to you the method of dividing "sexagesimal fractions". | ואחר שהתבאר זה ראוי שנבאר לך אופן החלוק לפי שברי חכמי התכונה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| You already know that the result of multiplying fractions by fractions belongs to the rank whose distance from [the rank of] the multiplier is the same as the distance of the multiplicand from the rank of units. | וכבר ידעת שהכאת שברים על שברים הוא מהמעלה אשר מרחקה מהמכה לפניה כמרחק המוכה ממעלת האחדים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| With that understood, I will give you a way of multiplying integers and fractions; from this you can know all the ways of multiplying fractions. | וכאשר התישב זה אתן לך דרך לכפול שלמים ושברים ומזה תוכל לדעת אופני הכפלת שברים בכל אופניהם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| When you want to multiply integers and fractions of the sexagesimal fractions by integers and fractions of this type, we should also write down the number that has fewer ranks in one row according to its ranks, and make a sign between the integers and the fractions in the way we mentioned above. | כאשר תרצה לכפול שלמים ושברים משברי חכמי התכונה על שלמים ושברים משבריהם ג"כ ראוי שנכתוב המספר אשר אחז יותר מעט מהמדרגות בטור אחד כפי מדרגתו ותעשה רושם בין השלמים והשברים על הדרך אשר זכרנו במה שקדם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Then, write down the other number in another row beneath it according to its rank. | ואחר תכתוב המספר האחר בטור אחד תחתיו כפי מדרגותיו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Multiply the first number of the upper row by the first of the bottom row and write the result in the appropriate rank. | ותכה הראשון שבטור העליון על הראשון שבטור התחתון ותשים העולה במעלה הראויה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If the result is more than sixty, divide the result by sixty; what you receive are units in the next rank. Write the remainder in the appropriate rank. | ואם עלה יותר מששים תחלוק העולה על ששים והעולה בידך יהיו אחדים במעלה שלאחריו והנשאר תשים במעלה הראויה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If the result is more than sixty in the next rank, you divide the result again by sixty. | ואם עלה יותר מששים במעלה שלאחריה תשוב לחלק העולה על ששים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| And so on until you reach the rank of units, and from there on you only divide by ten. The reason for this is obvious. | וכן עד הגיעך למעלות האחדים ומשם והלאה לא תחלוק כי אם על עשרה והסבה מבוארת | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| You continue like this until all the numbers of the upper row have been multiplied by all the numbers in the bottom row. | וכזה תעשה עד שיוכו כל מספרי הטור העליון על כל מספרי הטור התחתון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| There are then as many rows of results as the number of ranks in the upper row, in which there are numbers, except for the integers, for which there is only one row [of results], however many they may be. | ויהיו טורי העולה כמספר המדרגות שבטור העליון אשר בהם מספר זולת השלמים שלא יהיה להם כי אם טור אחד כמה שיהיו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Then, you add up all that is in the rows of results in a bottom row beneath them and the result is the required. | עוד תחבר כל מה שבטורי העולה בטור שפל תחתיהם והעולה הוא המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| When you get to multiply the fractions by the integers, multiply them together by all the integers of the bottom row at once, so that it does not confuse you, then divide the result by sixty as above, and write the remainder each time in the proper place. | וכאשר תגיע להכות השברים על השלמים תכה אותם יחד על כל השלמים שבטור התחתון כדי שלא יתבלבל עליך ותחלק העולה על ששים על הצד הקודם ותשים הנשאר כפעם בפעם במקום הראוי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| When you get to multiply the integers by the fractions, multiply all the integers that are in the upper row together as well by the fractions of the bottom row each time. | וכן כשתגיע להכות השלמים על השברים תכה גם כן כל השלמים שבטור העליון יחד על השברים שבטור התחתון כפעם בפעם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה רצינו בזאת הצורה להכות נ"ז שניים וט' ראשונים ופ"ג שלמים על שבעת אלפים ותשעים ומ' שניים ונ"א שלישיים וג' רביעיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הכינו נ"ז שניים על ג' רביעיים ועלה ב' חמשיים ונ"א ששיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הכינו נ"ז שניים על נ"א שלישיים ועלה מ"ח רביעיים וכ"ז חמשיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הכינו נ"ז שניים על מ' שניים ועלה ל"ח שלישיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הכינו נ"ז שניים על ז' אלפים וצ' שלמים ועלה ל' שניים ט"ו ראשונים וב' בראשונה א' בשנייה א' בשלישית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר הנהגו בכמו זאת ההנהגה עד שיוכו כל מספרי הטור העליון על מספרי הטור התחתון תמצא העולה נ"א ששיים נ"ו חמשיים ל"ו רביעיים כ"ג שלישיים ז' שניים ל"ב ראשונים ה' שלמים ד' ו' ט' ח' ה' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Deduce from this. | והקש על זה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Division of sexagesimal fractions |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If you wish to divide a number by integers and fractions of the fractions that we are dealing with, write the number you wish to divide in an upper row in its positions, so that you will be able to write the result between these two rows as you did previously. | ואם תרצה לחלק מספר מה על שלמים ושברים מאלה השברים אשר אנחנו בהם תכתוב המספר שרצית לחלק בטור העליון במקומותיו בדרך שתוכל לכתוב העולה בין שני אלו הטורים כמו שעשית במה שקדם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Then look what is in the last rank of the bottom row, consider them as units. | אחר כך תראה מה שבמעלה האחרונה שבטור התחתון והיו לאחדים בידך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Consider all the numbers that precede the preceding rank as one in the preceding rank. | וכל המספרים אשר לפני המעלה הנמשכת לה לפניה תחשוב אחד במעלה הנמשכת לה לפניה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Add it to the number that is in it. | ותחברהו עם המספר שבה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If the rank before the last contained integers, consider this number as tenths. | ואם היתה זאת המעלה שלפני האחרונה שלמים יהיה זה המספר עשיריות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If this rank before the last contained fractions, this number will be sixtieths. | ואם היתה זאת המעלה שלפני האחרונה שברים יהיה זה המספר חלקים מששים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Add it to the units that are in the last rank and this is the reserved by which you divide the last rank of the upper row with what is in the preceding rank, be this tenths or sixtieths. | וחברהו עם האחדים שבמעלה האחרונה והוא השמור ועליו תחלוק המעלה האחרונה שבטור העליון עם מה שבמעלה לפניה אם עשיריות ואם חלקים מששים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Place the result in the appropriate rank according to the above and multiply is by the bottom row, subtract the result from the upper row, and proceed so until you are left with less than the row by which you have divided [= the divisor]. | והעולה תשים במעלה הראויה לפי מה שקדם וערכהו על הטור התחתון והעולה גרע מהטור העליון וכן תעשה עד שישאר לך פחות מהטור שחלקת עליו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה רצינו שנחלוק ז' מאות ומ' ראשונים ונ' שניים על ט' שלמים וכ' ראשונים ול' שלישיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה נחשוב המספרים שלפני המעלה הנמשכת לאחרונה שבטור התחתון אחד בה וכ' שמצאנו בה והנה כ"א והם חלקים מששים ובמעלה האחרונה ט' והם אחדים והנה השמור הוא ט' אחדים וכ"א חלקים מששים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקנו על השמור המספר שבמעלה האחרונה שבטור העליון שהוא שבעים אחר שהורדנוהו אל המעלה שלפניה ועלה ז' מהמעלה השניה לפי מה שקדם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונכתוב ז' בטור האמצעי במעלה השניה ונערכהו על הטור התחתון וכאשר הנהגנו זה על הצד שזכרנו תמצא העולה ז' מהמעלה השניה ה' מהמעלה הראשונה ונשאר בטור העליון שלא הגיע לחלוק מ' ראשונים י"ב שניים ל' שלישיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Deduce from this. | והקש על זה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The way of dividing what cannot be divided when there are integers in the bottom row. | דרך לחלק מה שלא הגיע לחלוק כשהיו בטור התחתון שלמים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| We suggest for this explanation that the division of any fractions by integers results in fractions of the same rank. | ונציע לביאור זה שחלוק שברים אי זה שיהיו על שלמים הם שברים מהמעלה ההיא בעינה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| This is clear from the multiplication. | וזה מבואר מצד הכפל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| When this is clear to you, look how many primes you have left in the upper row when you convert the last [rank] of the upper row to the rank of the primes. | וכאשר התישב לך זה הנה תראה כמה מן הראשונים נשארו לך בטור העליון כשתשיב האחרון שבטור העליון למדרגת הראשונים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Consider the number that you have of the primes as units and what is in the preceding rank is sixtieths. | והמספר ההוא שיהיה לך מן הראשונים יהיו לאחדים בידך ומה שבמדרגה שלפניה יהיו חלקים מששים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Divide the result by the reserved; the result is primes, write them in the row of the result in their positions. | והעולה תחלוק על השמור והעולה בידך הם ראשונים כתבם במקומותם בטור העולה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Multiply them by the whole bottom row, by which you divide, then subtract the result from the upper row. | וערכם על כל הטור התחתון שתחלוק עליו והעולה תגרע מהטור העליון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Divide the last [rank] of the upper row by the reserved. | עוד תחלוק האחרון שבטור העליון על השמור | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If you cannot divide it, lower it to the preceding rank. | ואם לא תוכל לחלק תורידהו אל המדרגה שלפניו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| You can calculate is more precisely endlessly. | ובזה הדרך תוכל לדקדק עד אין קץ | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| However, the way to find the reserved is by lowering all the integers that are in the bottom row to the first rank, considering the result as units and everything that is precedes the first rank as sixtieths, according to the previous way. | ואולם דרך לקיחת השמור הנה יהיה שתוריד כל מה שבטור התחתון מן השלמים אל המעלה הראשונה והעולה יהיה לאחדים בידך ומה שלפני המעלה הראשונה יהיו חלקים מששים על הצד הקודם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| This is your way to find the reserved, for what cannot be divided when there are integers in the bottom row. | וזה יהיה לך דרך לקיחת השמור במה שלא הגיע לחלוק כאשר היו בטור התחתון שלמים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה במה שנשאר במשל הקודם שלא הגיע לחלוק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקנו מ' וי"ב חלקים על השמור שהוא ט' וכ"א מששים ועלה ד' חלקים והם ראשונים מפני שחלקנו ראשונים על שלמים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ערכנו ד' ראשונים על הטור התחתון ועלה ל"ז ראשונים כ' שניים ב' שלישיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וגרענו זה מהטור העליון ונשאר שם ב' ראשונים נ"ב שניים כ"ח שלישיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולא נוכל לחלק ב' ראשונים על השמור הורדנום אל השניים והנה קע"ב וכ"ח חלקים מששים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקנום על השמור וגרענו העולה מהטור העליון ונשאר שם ד' שניים כ"ח שלישיים נ"א רביעיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| This way you can approximate further to thirds and fourths and other, but there is no need to approximate further, since you receive an approximate result. | ובזה הדרך תוכל לדקדק עוד לשלישיים ורביעיים ולזולתם ואין צורך לדקדק אחר שתגיע אל החשבון בקירוב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The method of division, when the last rank of the lower row is a rank of fractions: | דרך החלוקה כאשר היתה המדרגה האחרונה שבטור התחתון ממדרגת השברים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Know that [the result of] division of fractions by fractions of the same kind is integers, and [the result of] division of fractions of a higher rank is of a rank, whose distance from the integers is the same as the distance of the divided fractions from the fractions by which they are divided. The reason is evident from the above. | דע כי חלוק שברים על שברים ממינם הוא שלמים וחלוק שברים יותר גבוהים מהם הוא מהמדרגה אשר מרחקה מהשלמים לפניה כמרחק השברים המחולקים מהשברים אשר חולק עליהם והסבה מבוארת ממה שקדם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה אם נחלק שניים על שניים יהיה העולה שלמים ואם נחלק שלישיים על ראשונים יהיה העולה שניים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Deduce from this. | והקש על זה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If you wish to divide any number by any number, and the last rank of the row of the divisor is of the ranks of fractions, look at the last rank of the upper row [= the dividend] - if it is higher than the last rank of the bottom row, lower it to the rank of the bottom row so that the last rank of the upper row is the same as the last rank of the bottom row. | אם רצית לחלק מספר מה על מספר מה והיתה המדרגה האחרונה שבטור שתחלוק עליו ממדרגת השברים הסתכל על המדרגה האחרונה שבטור העליון אם היא יותר גבוהה ממדרגה האחרונה שבטור התחתון אז תורידהו אל המדרגה שבטור התחתון עד שתהיה המדרגה האחרונה שבטור העליון היא בעינה המדרגה האחרונה שבטור התחתון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Then divide the last [rank] of the upper row with the sixtieths fractions that precede it, according to the previous way, by the reserved; the result will be integers. Multiply them by the bottom row and subtract the result from the upper row. Divide also what remains in the last rank of the upper row by the reserved and place the result in the corresponding rank. Multiply it by the bottom row and subtract the result from the upper row. Proceed like this until there is nothing left for you in the upper row or only a little is left for you there. | ואז תחלק האחרונה שבטור העליון עם החלקים מששים אשר לפניה על הצד הקודם על השמור והעולה יהיו שלמים ותערכם על הטור התחתון ותגרע העולה מהטור העליון עוד תחלק הנשאר במעלה האחרונה שבטור העליון על השמור והעולה תשים במעלה הראויה ותערכהו על הטור התחתון ותגרע העולה מהטור העליון וכן תעשה עד שתגיע שלא ישאר לך בטור העליון דבר או שיהיה מעט מה שישאר לך שם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה רצית לחלק שבעה עשר שלמים ול' ראשונים ומ' שניים על מ"א שניים ונ"ב שלישיים ומ"ה רביעיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולפי שהמעלה האחרונה שבטור התחתון היא שניים נוריד מה שאחר השניים בטור העליון אל מדרגת השניים ולזה נוריד השבעה עשר אל מדרגת הראשונים ויהיו אלף וכ' ול' שהיו שם והנה אלף ונ' ראשונים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הורדנום אל מדרגת השניים ועלה ס"ג אלפים ומ' שניים ואלה יהיו לאחדים בידך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה מה שבמדרגה האחרונה שבטור התחתון הוא מ"א ונחשבו כמו אחדים ומה שלפניהם הוא לפי מה שקדם נ"ג חלקים מששים ולזה יהיה השמור מ"א ונ"ג חלקים מששים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקנו ס"ג אלפים ומ' על מ"א ונ"ג חלקים מששים ועלה אלף וחמש מאות וחמשה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ערכנו אלף ותק"ה על הטור התחתון ועלה י"ז שלמים ל' ראשונים כ"ח שניים ח' שלישיים מ"ה רביעיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
גרענו העולה מהטור העליון ונשאר י"א שניים נ"א שלישיים ט"ו רביעיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולא נוכל לחלק מה שבמדרגה האחרונה שבטור העליון על השמור ולזה נוריד אל השלישיים ויהיו לנו תשי"א וט"ו חלקים מששים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקנום על השמור ועלה י"ו והם ראשונים לפי מה שקדם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ערכנו י"ו ראשונים על הטור התחתון וגרענו העולה מהטור העליון ונשאר בטור העליון מ"א שלישיים י"א רביעיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולא נוכל לחלק מ"א וי"א חלקים מששים על השמור ולזה נוריד השלישיים על הרביעיים ויהיו לנו אלפים תע"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונחלקם על השמור ועלה נ"ח והם שניים לפי מה שקדם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ערכנום על הטור התחתון וגרענו העולה מהטור העליון ונשאר בטור העליון ל' שלשיים מ"ב רביעיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה נוכל לחלק האחרון שבטור העליון על השמור ועלה אחד והוא שני לפי מה שקדם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ערכנוהו על הטור התחתון וגרענו העולה מהטור העליון ונשארו בטור העליון ז' חמשיים מ"ה ששיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תרצה תוכל לדקדק עוד ולהוריד חמשיים אל הששיים ולחלק על השמור ויהיה העולה רביעיים לפי מה שקדם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואין צורך כי כבר הגענו אל קירוב גדול | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Here the explanation of the division of a number by a number in all the dividing methods is complete. | ובכאן נשלם הביאור בחלוק מספר על מספר בכל אופני החלוקה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Dividing a known number by an unknown number |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Since the way of dividing a known number by a known number has been explained, we should explain the way of dividing a known number by an unknown [number], as in the extraction of the square and cube roots of given numbers. | ואחר שהתבאר דרך חלוק מספר ידוע על מספר ידוע ראוי שנבאר דרך חלוק מספר ידוע על מספר בלתי ידוע כמו הוצאת השרשים הרבועיים והמעוקבים ממספרים המונחים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Extraction of Square Roots |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| First, we shall explain the way of extracting the square roots. | ונבאר תחלה דרך הוצאת השרשים הרבועיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| We base the explanation on the theorem that it is impossible to find roots of integers whose root is not an integer. | ונציע לביאורו הביאור שא'א' שימצא יסוד מספרי למספרים המקיפים בשלמים שאין יסודם אחדים שלמים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| This is because one is a square number. | וזה שהאחד הוא מרובע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| But, you already know from Book VIII of Euclid, proposition 14, that when a square measures a square, its side measures the side [of the other]. | וכבר ידעת מח' מאקלידס י"ד שכאשר ימנה מרובע מרובע הנה צלעו ימנה צלעו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Since, one measures every number, even if this number is a square, then one must also measure its root, but if one does not measure it, then the number cannot be a square number. | והאחד ימנה כל מספר ואם היה זה המספר מרובע הנה האחד מונה את יסודו אבל האחד לא ימנהו אם כן אין מספר מרובע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Therefore, it has been proven that this number cannot have a root. | ולזה יתבאר שא'א' שיהיה לזה המספר יסוד מספרי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
משל זה שמספר העשרה אין יסודו מקיף בשלמים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
לפי שמרובע שלשה הוא תשעה ומפני שהעשרה מוסיף על תשעה יהיה יסודו מוסיף על יסודו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ובזה יתבאר שיסוד עשרה הוא פחות מארבעה לפי שמרובע ארבעה הוא ששה עשר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
א"כ אין יסוד עשרה מקיף בשלמים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה עשרה ימנהו האחד שהוא מרובע ואם היה עשרה מרובע היה יסוד העשרה ימנהו יסוד האחד שהוא אחד וזה כבר התבאר שהוא שקר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם כן אין למספר עשרה יסוד מספרי לא נשבר ולא בלתי נשבר ולזה יקרא יסודו הוא מדבר בכח לבד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Deduce from that. | והקש על זה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The ranks of the square numbers | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| This being understood, we shall teach you in which rank one can extract the square root, and in which ones it cannot. | וכאשר התישב זה נודיעך אי זה מהמדרגות יתכן שילקח השרש מהם ואי זה מהם לא יתכן לו בם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Know that the squares of the numbers from one to ten are: 1; 4; 9; 16; 25; 36; 49; 64; 81. | דע כי מרובעי המספרים הנמשכים מן האחד עד עשרה הם מספר א' ד' ט' ששה עשר כ"ה ל"ו מ"ט ס"ד פ"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| As the units of the ranks are proportional, starting from one, and the second, which is ten, is not a square number, then there are square numbers only in the first [rank], the third, the fifth, and so on for all odd ranks. | ולפי שאחדי המעלות מתיחסים ומתחילין מן האחד והשני שהוא עשרה בלתי מרובע הנה אין שם אחד מרובע אלא הראשון והשלישי והחמשי וכן כל המעלות הנפרדות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| This being understood, it is clear that every square number which is in an odd rank is a square number, because it is counted by the unit of this rank, which is a square number, as the number of units of a square number. | וכאשר התישב זה התבאר שאי זה מספר מרובע שימצא במעלות הנפרדות הוא מרובע לפי שהמספר ההוא ימנה האחד מזאת המעלה שהוא מרובע כמספר אחדי מספר מרובע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| So, the result is a product of a square number by a square number, which is again a square number according to the above. | אם כן העולה הוא שטח מספר מרובע במספר מרובע שהוא מרובע לפי מה שקדם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Likewise, it is clear that any square number that is in an even rank cannot possibly be a square number. | ובכמו זה התבאר שאי זה מספר מרובע שימצא במעלות שהם זוג אי אפשר שיהיה מרובע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Also, it is clear concerning the ranks of sexagesimal fractions that the units of every even rank are square numbers, whereas the units of the odd ranks are not square numbers. | וכזה הת' במעלות השברים משברי חכמי התכונה שכל מעלה שהיא זוג אחדיה מרובעים והמעלות הנפרדות אין אחדיהן מרובעים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| This is because sixty is not a square, therefore a prime cannot be a square, because it counts one, which is a square, by the number sixty, which is not a square, so a prime is not a square. | וזה שמספר הששים איננו מרובע ובהיות הענין כן יתחייב שלא יהיה השבר הראשון מרובע לפי שהוא ימנה האחד והוא מרובע במספר ששים והוא בלתי מרובע אם כן אין השבר הראשון מרובע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| I claim that a second is square, because the ratio of one to a prime is the same as the ratio of a prime to a second, so the product of a prime by itself is the same as the product of one by a second, but the product of one by a second is a second, so a second is a square number and its root is a prime. | ואומר שהשבר השני מרובע וזה שיחס האחד אל הראשון כיחס הראשון אל השני א"כ שטח הראשון בעצמו כמו שטח האחד בשני אבל שטח האחד בשני הוא שני אחד אם כן השני מרובע ויסודו הראשון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| It is clear from this a fourth is a square, because the ratio of one to a second is the same as the ratio of a second to a fourth, so the product of one by a fourth is the product of a second by itself, therefore a fourth is a square. | ובזה התבאר שהרביעי מרובע וזה שיחס האחד אל השני כיחס השני אל הרביעי א"כ שטח האחד ברביעי הוא שטח השני בעצמו אם כן הרביעי מרובע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Likewise it is clear that all even ranks are square numbers. | ובזה התבאר שכל מעלות הזוגות הם מרובעות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| And so it is clear that every number that is in an even rank must be a square number, because its units are square numbers. | ולזה התבאר שאי זה מספר שיהיה במעלות אשר הם זוגות הוא מרובע לפי שאחדיה מרובעים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| It is also clear from this, the way Euclid proved it, that because one is a square number, the third from it of the proportional units must be a square number, therefore the second is a square number, and the fourth is a square number and so are the even ranks that follow. | ובזה יתבאר זה גם כן בצד הביאור אשר באר אקלידס כי האחד לפי שהוא מרובע השלישי לו מהאחדים המתיחסים הוא מרובע ולזה יהיה השני מרובע והרביעי מרובע ומה שימשך מזה מהמעלות הזוגות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The way of extracting the square root of integers | דרך הוצאת השרש מהמספר המרובע המקיף בשלמים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| We should write down the number, whose square root we are looking for, in a row, arranged according to its ranks. | ראוי שנכתוב המספר שבקשנו לדעת את מרובעו בטור אחד כפי מעלותיו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Then, examine the last rank in the row, whether it is odd or even: | אחר כך חקור על המעלה האחרונה שבטור אם היא מהנפרדות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If it is not odd, lower it to the preceding [rank], so that the last number is in an odd rank. | ואם לא היתה מהנפרדות הורידה אל שלפניה כדי שיהיה המספר האחרון שבמעלה נפרד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Then, look for the square number that is the closest to that number, but smaller. You write the root of that square in the row of the root, beneath the previous row in the rank that is mean between the first rank and the last rank - this is the row that we call the result row. | אחר כך ראה המרובע היותר קרוב אל זה המספר ואמנם המעט ויסוד המרובע ההוא תכתוב בטור השרש תחת הטור הקודם במעלה האמצעית בין המעלה הראשונה והמעלה האחרונה והוא אשר נקראה הטור היוצא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Subtract the square of the resulting root from the top row and divide the remainder by twice the resulting root. | ומרובע השרש היוצא תגרע מהטור העליון והנשאר תחלוק על כפל השרש היוצא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| But, make sure that after the division you are left with as much as the square of the root resulting from division and the result of division, and this is the resulting root. | אך השמר שישאר לך אחר החלוקה כמו מרובע השרש היוצא לך מן החלוקה והעולה בחלוק והוא השרש היוצא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Write it in the row of the root, in the rank that is as far backward from the rank that you divided as the rank by which you divided is far from the first [rank]. | תכתבהו בטור השרש במעלה אשר מרחקה לאחור מהמעלה שחלקת כמרחק המעלה שחלקת עליה מהראשונה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| ותערוך זה השרש היוצא לך מן החלוקה והעולה בחלוק תכתבהו בטור השרש במעלה הראויה מצד הקודם | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Multiply the root that resulted from the division by double the found root and by itself and subtract the result from the top row. | והשרש היוצא מן החלוקה תערוך על כפל השרש המוצא ועל עצמו והעולה תגרע מהטור העליון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Continue this way until nothing is left in the upper row. | וכן תעשה עד שלא ישאר בטור העליון דבר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה אם רצית להוציא שרש א'ח'ב'ו'ד'ו'ב'ח' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולפי שהמעלה האחרונה היא שמינית תורידה אל שלפניה והנה פ"ב והנה פ"א הוא המרובע היותר קרוב לזה המספר ושרשו ט' תכתוב ט' בטור השרש ברביעית שהיא אמצעית בין השביעית והראשונית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה מרובע ט' מהרביעית הוא פ"א מהשביעית גרענום מפ"ב ונשאר אחד בשביעית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולא נוכל לחלק על כפל ט' שהוא השרש המוצא הורדנוהו אל שלפניו עם ו' שהיו בה והנה י"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולא נוכל לחלק על כפל ט' הורדנו הי"ו אל שלפניה והנה קס"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקנום על כפל השרש המוצא שהוא י"ח ועלה ט' והוא השרש היוצא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונכתבם בטור השרש ברביעית לאחור במדרגת קס"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ערכנום על כפל השרש המוצא ועל עצמם והעולה גרענו מהטור העליון ונשאר בו א'ח'א'ח'א' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקנום על כפל השרש המוצא והוא על צד הקודם י"ח אחדים וב' עשיריות ועלה אחד בראשונה בקרוב ונכתבהו בראשונה בטור השרש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ערכנוהו על כפל השרש המוצא ועל עצמו וגרענו העולה מהטור העליון ולא נשאר בטור העליון דבר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה שרש זה המספר הדרוש הוא ט' אלפים וצ"א והוא המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תרצה תוכל לבחון זה בשתכה טור השרש על עצמו ויצא לך הטור העליון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והיה זה כן לפי שכבר התבאר שכאשר הוסף מספר על מספר הנה מרובע שני המספרים מקובצים שוים למרובעי שני המספרים ולכפל שטח זה בזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Extracting root of integers and simple fractions | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The way of extracting the roots of square numbers that are not compound of whole units and in which there are no sexagesimal fractions. | דרך הוצאת השרשים מהמספרים המרובעים אשר הם בלתי מקיפים באחדים שלמים ולא היו השברים בהם משברי חכמי התכונה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Find the common denominator of these fractions, i.e. the least common multiple of the denominators of all these fractions. | הוצא המורה הראשון אל השברים ההם ר"ל המספר המעט שימנה מורי השברים ההם בכללם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Multiply that number by itself, if it is a square number, or by its square, if it is not. Then, extract the root of the result and divide it by the square root of the number by which you multiplied the given number; the result is the required. | ועליו אם היה מרובע או על מרובעו אם לא היה המורה הראשון מרובע תערוך המספר ההוא ותוצא שרש העולה וחלקהו על שרש המספר אשר כפלת עליו המספר המונח והעולה הוא המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון אם רצית לדעת שרש פ"ב שלמים ורביע וב' שביעיות שביעית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה המורה הראשון לאלו השברים הוא לפי מה שהתבאר מאקלידס קצ"ו והוא המספר המורכב מד' ומ"ט שהם מרובעים ולזה יהיה קצ"ו מרובע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ערכנו עליו זה המספר ועלה ט'ב'א'ו'א' לקחנו שרשו ועלה קכ"ז חלקנוהו על שרש קצ"ו שהוא י"ד ועלה ט' וחצי שביעית וככה המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והקש על זה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והיה זה כן לפי שכבר התבאר שכאשר הוכה מספר מרובע במרובע שיסוד העולה הוא המספר המורכב מיסודי שני המרובעים אם כן העולה ימנה היסוד האחד כמספר אחדי יסוד האחר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The way to extract the square root of non-square numbers with great approximation when there are integers in the root | דרך להוצאת שרש מספר בלתי מרובע שיהיה בשרשו שלמים בקירוב גדול | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| First, extract the square root that is nearest to [the true root of] that number in the way we have mentioned, so that in the upper row you are left with less than double the found root plus one, which is the square of the resulting root. | הוצא תחלה השרש הקרוב למספר ההוא בדרך שזכרנו עד שישאר לך בטור העליון פחות מכפל השרש המוצא מקובץ עם אחד שהוא מרובע השרש היוצא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Lower what you are left with down to [the rank of] primes and divide by twice of all that is in the row of the root, when you lower it down to the rank of units. But, make sure you are left with the square of the resulting root in the upper row. The result are primes according to the above. | והנשאר לך תורידהו אל הראשונים וחלק על כפל כל מה שבטור השרש כשתורידהו למעלת האחדים והזהר שישאר לך בטור העליון כמו מרובע השרש היוצא והעולה יהיו ראשונים לפי מה שקדם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Multiply them by double the found root and by themselves, then subtract the result from the upper row. | ערכם על כפל השרש המוצא ועל עצמם וגרע העולה מהטור העליון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If the remainder is less than double the found root plus one, lower it down to the preceding rank and divide again by double the found root, after you have lowered all the integers down to the first rank and the fractions that precede them are considered as parts of sixty. | והנשאר לך אם הוא פחות מכפל השרש היוצא נחבר עם אחד תורידהו אל המעלה שלפניה ותשוב לחלק על כפל השרש המוצא כשתוריד כל השלמים אל המעלה הראשונה והשברים אשר לפניהם יהיו חלקים מששים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The resulting square root is, according to the above, of the rank of the fractions we divided; write it there in the row of the result. | והשרש היוצא הוא לפי מה שקדם ממעלת השברים אשר חלקנו ושם תכתבהו בטור העולה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Multiply it by double the found square root and by itself, then subtract the result from the upper row. | ערכהו על כפל השרש המוצא ועל עצמו והעולה גרע מהטור העליון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| This way you can approximate as much as you want. | ובזה הדרך תוכל לדקדק כפי מה שתרצה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה אם רצית למצא שרש מספר א'ב'ג'ד'ה'ו'ז' ומ' ראשונים ול' שניים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה תוצא השרש הקרוב על הצד הקודם ויעלה ו'ו'ז'ב' ונשאר בטור העליון ג' אלפים וה' מאות וס"ה שלמים מ' ראשונים ל' שניים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הורדנו השלמים למדרגת הראשונים ויהיו בידינו מאתים אלף וי"ג אלפים וט' מאות ומ' ובמדרגה שלפניהם ל' והם חלקים מששים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקנו העולה על כפל הטור התחתון שהוא ה' אלפים וה' מאות ול"ב בדרך שישאר כמו מרובע השרש היוצא ועלה ל"ח והם ראשונים לפי מה שקדם ושם נכתבם בטור השרש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ערכנום על כפל השרש המוצא ועל עצמם וגרענו העולה מהטור העליון ונשאר בטור העליון ס"א שלמים מ' ראשונים כ"ו שניים שהם ג' אלפים ות"ש ראשונים וכ"ו שניים וזה פחות מכפל השרש המוצא שהוא ה' אלפים וה' מאות ול"ג שלמים וי"ו חלקים מששים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הורדנו הנשאר בטור העליון למדרגת השניים ויהיו בידינו מאתים אלף וכ"ב אלפים וכ"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקנום על כפל השרש המוצא ועלה מ' והם שניים לפי מה שקדם ושם נכתבם בטור השרש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ערכנום על כפל השרש המוצא ועל עצמם וגרענו העולה מהטור העליון ונשאר בטור העליון י"א ראשונים ל"ד שניים נ"ג שלישיים כ' רביעיים שהם תרצ"ד שניים נ"ג שלישיים כ' רביעיים וזה פחות מכפל השרש המוצא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולזה נוריד כל זה למדרגת השלישיים יעלה מ"א אלפים ותרצ"ג וכ' חלקים מששים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקנום על כפל השרש המוצא שהוא ה' אלפים ה' מאות ול"ג וי"ח חלקים מששים ועלה ז' שלישיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ערכנום על כפל השרש המוצא ועל עצמם וגרענו העולה מהטור העליון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומצאנו שהגענו אל השרש הדרוש בקירוב גדול כי הנשאר בטור העליון אינו מגיע לשני אחד והוא מעט כשיוקש אל מה שראוי שיתחלף השרש האמתי בעבורו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תרצה תוכל לדקדק עוד ואין צורך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Another way when there are integers in the root | דרך אחרת כשהיה בשרש שלמים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Know that the larger the number whose root you want to know, the harder it is to find that root. | דע כי כל אשר יהיה המספר שתבקש לדעת שרשו יותר גדול תהיה הוצאתו יותר בקושי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| I will show you how to turn a large number into a smaller one, after I explain to you that the multiplication of a number by 36 seconds equals its division by a hundred: | ואודיעך איך תעשה ממספר גדול מספר מעט אחר שאבאר לך כי כפל מספר בל"ו שניים הוא שוה לחלוקו אל מאה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| This is so because 36 seconds is one part of a hundred of a unit, since there are 36 hundred seconds in a unit.
|
וזה כי ל"ו שניים הוא חלק אחד ממאה באחד כי השניים אשר באחד הם ל"ו מאות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| When that has become clear to you, divide the large number by a hundred. | וכאשר התבאר לך זה הנה תחלוק על מאה המספר הגדול | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| This division is very easy as to what can be divided. | וזה החלוק יקל מאד במה שיגיע ממנו לחלוק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Regarding what cannot be divided: multiply it by 36 seconds; the integers and fractions you receive are the larger number lowered once. | ומה שלא יגיע ממנו לחלוק תערכהו על ל"ו שניים והעולה בידך מהשלמים והשברים הוא המספר הגדול מורד פעם אחת | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If this lowered number is not less than a hundred, lower it again in this way until it becomes less than a hundred. | ואם לא היה זה המספר המורד פחות ממאה תשוב להוריד אותו בזה הדרך עד שיגיע פחות ממאה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The last lowered number is the number whose square root is required. | והמספר האחרון המורד הוא המספר המבקש יסודו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| You can find it very easily and approximate it to fifths or thirds, so that the calculation will reach an extreme approximation. | והנה תמצאהו בקלות גדול ותדקדק עד חמשיים או עד שלישיים כדי שיהיה החשבון בקירוב מופלג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| When you find it, count the number of lowerings and take the number composed of as many tens as the number of lowerings. This corresponds to the rank, whose positional value exceeds the number of lowerings by one. | וכאשר תמצאהו תמנה מספר ההורדות וקח המספר המורכב ממספרי עשרה כמספרי ההורדות וזה מסכים למעלה אשר מספרה מוסיף אחד על מספר ההורדות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Multiply the square root you have by the result, and the result of the multiplication is the required. | ועל העולה ערוך השרש שיש לך ומה שיגיע מן הכפל הוא המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון רצינו שנוציא שרש א'ב'ג'ד'ה'ז'ח'ט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקנו זה המספר על מאה וערכנו הנשאר שלא בא לחלוק על ל"ו שניים ויצא לנו ג'ד'ה'ז'ח'ט' וי"ב ראשונים ל"ו שניים וזאת היא ההורדה הראשונה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולפי שמה שיצא לנו הוא בלתי קטן ממאה נשוב לחלק זה הטור שיצא לנו על מאה ולערוך מה שלא בא לחלוק על ל"ו שניים ויצא לנו ה'ז'ח'ט' וכ"ה ראשונים נ"ה שניים ל"ג ל"ו והיא ההורדה השנית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונשוב עוד לחלק זה הטור שיצא לנו על מאה על הדרך הקודם ויצא לנו ח' בראשונה ט' בשניה מ"ה ראשונים ט"ו שניים ל"ג שלישיים כ' רביעיים ט' חמשיים ל"ו ששיים וזה המספר הוא קטן ממאה ולזה לא נשוב להוריד עוד והנה ההורדות שלשה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה נחקור על שרש זה המספר הקטן | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולפי שהמעלה האחרונה שבטור היה מהזוגות נוריד אל שלפניו ויהיו לנו צ"ח בראשונה והנה המרובע הקרוב לזה המספר לפניו הוא פ"א ושרשו ט' מהראשונה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הורדנום אל מדרגת הראשונים ועלה אלף וס"ה וט"ו חלקים מששים על הצד הקודם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקנום על כפל השרש המוצא שהוא י"ח בדרך שישאר בטור העליון מרובע השרש היוצא ועלה נ"ו והם ראשונים לפי מה שהתבאר קודם ושם נכתבם בטור השרש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ערכנום על כפל השרש המוצא ועל עצמם וגרענו העולה מהטור העליון ונשאר בטור העליון ד' ראשונים נ"ט שניים ומה שנמשך להם מן השברים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הורדנו הראשונים למדרגת השניים והנה רצ"ט ול"ג חלקים מששים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקנום על כפל השרש המוצא שהוא י"ט שלמים ונ"ב חלקים מס' בדרך שישאר לנו מרובע השרש היוצא מן החלוקה ועלה ט"ו והם שניים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ערכנום על כפל השרש המוצא ועל עצמם וגרענו העולה מהטור העליון ונשאר לנו בטור העליון שני אחד כ"ט שלישיים ל"ה רביעיים ט' חמשיים ל"ו ששיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר נהגנו בכמו זה המנהג מצאנו זה השרש בקירוב גדול ט' שלמים נ"ו ראשונים ט"ו שניים ד' שלישיים ל' רביעיים כ"ו חמשיים נ"ב ששיים נ"ב שביעיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והקרוב היה לתוספת אצל המרובע ב' שביעיים מ"ב שמיניים ז' נ' ל' ל"ה ל"ח נ"ג ד' שמרהו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולפי שההורדות יהיו שלשה נערוך זה השרש על אלף כי המספר המורכב מג' דמיוני עשרה הוא אלף והנה העולה הוא ט' אלפים ותתקל"ח שלמים ל"ז ראשונים ט"ו שניים ז' שלישיים כ"ח רביעיים ג' חמשיים ו' ששיים מ' שביעיים והוא שרש המספר המבוקש בקירוב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולדעת הקירוב ערוך הקירוב הראשון ששמרת על מרובע אלף שערכת עליו השרש והנה הקירוב אל הצד שהיה אליו הקירוב הראשון ולזה יהיה הקירוב הראשון ט' רביעיים מ"ד חמשיים י"ח ששיים ל"ב שביעיים 0 ל"ה ל"ד ל"ד ד' כ"ו מ' וזה קירוב גדול לזה המספר הרב לפי שזה הקירוב איננו מגיע למרובע לרביע אחד מן השברים השלישיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והקש על זה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| This is so because the number of the first row is counted by the number of the lowered second row as the number of units in one hundred. So, if the second row is multiplied by one hundred, it becomes the same as the first row. | והיה זה כן לפי שמספר הטור הראשון ימנה הטור השני המורד במספר מה שבמאה מן האחדים אם כן הטור השני יוכה במאה ויהיה כמו הטור הראשון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| In the same way it is clear that the lowered last row multiplied by the [number] composed of three times one hundred, which is a thousand of a thousand, is the same as the first row. So, the ratio of the first row to the lowered last row is a thousand of a thousand. | וכזה הת' שהטור המורד האחרון יוכה במורכב משלשה דמיוני מספר מאה שהוא אלף אלפים ויהיה כמו הטור הראשון א"כ יחס הטור הראשון אל הטור האחרון המורד הוא אלף אלפים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Likewise, the ratio of the larger root to the smaller root is one thousand.
|
וג"כ הנה יחס השרש הגדול אל השרש הקטן הוא אלף | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Also the ratio of the square of the larger root to the square of the smaller root is a thousand of a thousand.
|
ויחס מרובע השרש הגדול אל מרובע השרש הקטן הוא אלף אלפים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Therefore, the ratio of the square of the larger root to the square of the smaller root is the same as the ratio of the first row to the last row. | ולזה יהיה יחס מרובע השרש הגדול אל מרובע השרש הקטן כיחס הטור הראשון אל הטור האחרון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| When we interchange [the terms], the ratio of the square of the larger root to the first row is the same as the ratio of the square of the smaller [root] to the last row. | וכאשר המירונו הנה יחס מרובע השרש הגדול אל הטור הראשון כיחס מרובע הקטן אל הטור האחרון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| But, the square of the smaller root is approximately the same as the last row. So, the square of the larger root is approximately the same as the first row. | אבל מרובע השרש הקטן הוא כמו הטור האחרון בקירוב אם כן מרובע השרש הגדול הוא כמו הטור הראשון בקירוב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Also, because the ratio of the square of the larger root to the first row is the same as the ratio of the square of the smaller root to the last row, and the square of the smaller root is greater than the last row, then the square of the larger root is greater than the first row. | וגם כן הנה מפני שיחס מרובע השרש הגדול אל הטור הראשון כיחס מרובע השרש הקטן אל הטור האחרון והיה מרובע השרש הקטן יותר גדול מהטור האחרון הנה מרובע השרש הגדול יותר גדול מהטור הראשון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| When we take the differences, the ratio of the square of the larger root to its excess over the first row is the same as the ratio of the square of the smaller root to its excess over the last row. | וכאשר הבדלנו הנה יחס מרובע השרש הגדול אצל יתרונו על הטור הראשון כיחס מרובע השרש הקטן אצל יתרונו על הטור האחרון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| When we interchange [the terms], the ratio of the square of the larger root to the square of the smaller root is the same as the ratio of the excess of the square of the larger root over the first row to the excess of the square of the smaller root over the last row. | וכאשר המירונו הנה יחס מרובע השרש הגדול אל מרובע השרש הקטן כיחס יתרון מרובע השרש הגדול על הטור הראשון אל יתרון מרובע השרש הקטן על הטור האחרון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| However, the ratio of the square of the larger root to the square of the smaller is a thousand of a thousand. So, the ratio of the excess of the square of the larger root over the first row to the excess of the square of the smaller root over the last row is a thousand of a thousand. | אבל יחס מרובע השרש הגדול אל מרובע השרש הקטן הוא אלף אלפים אם כן יחס יתרון מרובע השרש הגדול על הטור הראשון אל יתרון מרובע השרש הקטן על הטור האחרון הוא אלף אלפים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Deduce from this. | והקש על זה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The method of extracting the roots of sexagesimal fractions | דרך הוצאת השרשים משברים מונחים משברי חכמי התכונה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Examine the highest of all the ranks, if it is an even. | חקור על המדרגה הגבוהה מכלם אם היא מהזוגות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If it is not even, lower it down to the preceding [rank], so it becomes even. | ואם היא אינה מהזוגות הורידה אל שלפניה כדי שתהיה מהזוגות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| From the result extract the true or the approximate but smaller root and write it in the row of the root, in the rank that is halfway between that rank and the first [rank]. | ומהעולה הוצא השרש האמתי או הקרוב אמנם המעט ותשימהו בטור השרש במדרגה הממוצעת בין המדרגה ההיא ובין האחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If you have a remainder, lower it down to a lower rank, so that you can divide it by double the found root. | ואם ישאר לך תורידהו אל מדרגה שפלה עד שתוכל לחלקו על כפל השרש המוצא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Write the result of division in the rank whose distance backwards from the dividend rank is the same as the distance of the divisor rank from the first [rank]. | והעולה בחילוק תשים במעלה אשר מרחקה מהמדרגה המחולקת לאחריה כמרחק המדרגה שחלקת עליה מהראשונה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| This way you approximate as much as you like. | וכפי זה הדרך תדקדק כל מה שתרצה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה אם רצית להוצא שרש נ"ג שלישיים מ"א רביעיים נ' חמשיים כ"ה ששיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה המדרגה היותר גבוהה היא מדרגת השלישיים ואיננה מהזוגות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הורידה ויהיו בידך שלשת אלפים ורכ"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והשרש היותר קרוב לזה המספר הוא נ"ו ונכתבם במדרגת האמצעיים בין מעלת האחדים והרביעיים והם השניים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ערכנום על עצמם וגרענו העולה מהטור העליון ונשארו לנו פ"ה רביעיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נורידם אל החמשיים ויהיו בידנו ה' אלפים וק"נ חמשיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקנום על כפל השרש המוצא שהוא קי"ב בדרך שישאר לנו מרובע השרש היוצא ועלה מ"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולפי המעלה שחלקנו עליה היא שלישית למדרגת האחדים נשים המ"ה בטור השרש במעלה השלישית לאחור למעלה המחולקת ולזה יהיו אלו המ"ה שלישיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ערכנום על כפל השרש המוצא ועל עצמם וגרענו העולה מהטור העליון ונשאר בטור העליון ע"ו חמשיים מ' ששיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נוריד החמשיים אל הששיים ועלה ארבע אלפים ות"ר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקנום על כפל השרש המוצא שהוא קי"ג ול"ב חלקים מששים בקירוב ועלה ל"ג והם לפי מה שקדם חמשיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ערכנום על כפל השרש המוצא ועל עצמם וגרענו העולה מהטור העליון ונשאר בטור העליון נ"ד שביעיים ח' שמיניים ב' תשיעיים נ"א עשיריים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| You can approximate this way as much as you like. | וכזה תוכל לדקדק כל מה שתרצה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| But, there is no need to approximate further, since the approximation is accurate to less than one sixth. | ואין צורך לדקדק עוד הקרוב בלתי מגיע לששי אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Deduce from this. | והקש על זה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Extraction of Cubic Roots |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The way of extracting the cube roots | דרך הוצאת השרשים המעוקבים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| We offer its explanation that some numbers do not have cube roots. | ואנחנו מציעים לבאורו שקצת המספרים אין להם יסוד מספרי עקוביי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| This is so as it was already explained that when a cube counts [another] cube, its side counts its side. | וזה שכבר הת' שכאשר מעוקב ימנה מעוקב הנה צלעו ימנה צלעו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Therefore, it follows necessarily that for any integer number whose cube root is not an integer, it is impossible to find its cube root. | ולזה יחוייב בכל מספר מקיף בשלמים שאין יסודו אחדים שלמים שאי אפשר שימצא לו יסוד מספרי עקוביי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Because if it had a cube root, then it would have been a cube number; and since this number counts the one that is a cubic number, its side should count its side. | וזה שאם היה לו יסוד מספרי היה מספר מעוקב ולפי שזה המספר ימנה האחד שהוא מעוקב הנה צלעו ימנה צלעו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| However, if its side counts its side, its root would have been an integer, but it was assumed that its root is not an integer and this is absurd, so the number is not a cubic number. | ואם היה צלעו מונה צלעו היה יסודו מקיף בשלמים וכבר הונח יסודו בלתי מקיף בשלמים זה ישקר אם כן אין מספר מעוקב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Therefore, it is clear that neither the number 10 nor the number 60 is a cubic number, and that it is impossible to find them a cubic root. | ולזה יתבאר שאין מספר העשרה מעוקב ולא מספר הששים ושאי אפשר שימצא להם יסוד מספרי עקוביי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Since this is clear, and since the units of the ranks are proportional and begin with the one and the second, which is ten, is not a cubic number, then, no rank is cubic other than the fourth, the seventh and the tenth, and these are the ranks whose number minus one counts the three. | וכאשר התבאר זה והיו אחדי המעלות מתיחסים ומתחילים מן האחד והשני שהוא עשרה אינו מעוקב אם כן אין אחד מעוקב זולת הרביעי והשביעי והעשירי והם המדרגות שמספרם מונה שלשה כשחוסר מהם האחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Hence, it is clear that there is no rank among the ranks of the fractions that is cubic except the thirds, the sixths and the ninths, whose number counts the three. | ובזה התבאר שאין במדרגות השברים מעלה מעוקבת זולת השלישיים והששיים והתשיעיים שמספרם מונה שלשה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Also, the cubes of the successive number from one to nine are 1, 8, 27, 64, 125, 216, 343, 512, 729. | וג"כ הנה מעוקבי המספרים הנמשכים מן האחד עד תשעה הם א' ח' כ"ז ס"ד קכ"ה רי"ו שמ"ג תקי"ב תשכ"ט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Since this is so, it is clear that every cubic number that is found in a cubic rank is a cube, because it counts the one that is a cubic number as many times as a cubic number. | וכאשר היה זה כן הוא מבואר שכל מספר מעוקב שימצא במדרגה מעוקבת הוא מעוקב לפי שהוא ימנה האחד המעוקב ההוא במספר מעוקב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Hence, the cubic number is multiplied by a cubic number, so the result is necessarily a cubic number. | אם כן המעוקב כבר הוכה במעוקב ולזה יהיה העולה מעוקב בהכרח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| From this it is clear that a cubic number that is in a non-cubic rank is not a cube. | ובזה הת' שהמספר המעוקב במעלה בלתי מעוקבת הוא בלתי מעוקב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| As all this is established, we shall explain how to find the cube root of cubic integers or the approximation of the non-cubic numbers. | וכאשר התישב זה כלו הנה נבאר איך ימצא השרש העקובי למספרים המקיפים בשלמים המעוקבים או הקרוב לבלתי מעוקבים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| You should write down the number whose cubic root you want to know in one row according to its ranks. | ראוי שתכתוב המספר שרצית לדעת יסודו העקובי בטור אחד כפי מדרגותיו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Then check if the last rank is of the cubic ranks; if it is not, lower the number to the preceding number until it is in a cubic column. | אח"כ תראה אם המעלה האחרונה היא מהמעוקבות ואם לא הורד המספר לפניה עד שתהיה במעלה המעוקבת | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Examine the number that is in this cubic rank according to the cubic numbers that we have mentioned, and take the closest cubic number, but smaller, whose root is known to you; place this root in the row of the root in the rank that we will explaine to you. | והמספר שיהיה במעלה ההיא המעוקבת תחקור עליה במעוקבים שזכרנו ותקח המעוקב היותר קרוב אליו ואולם המעט ויסודו ידוע לך והיסוד ההוא תשימהו בטור השרש במעלה אשר נבאר לך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Divide the number, which indicates the position of this rank, by three, you are necessarily left with one, add it to the result of the division, and place the result in the rank whose number is so. | והוא שתחלוק מספר גובה המעלה ההיא על שלשה וישאר לך אחד בהכרח חברהו עם העולה מן החלוקה ובמעלה אשר מספרה ככה תשים העולה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
משל זה אם היתה המעלה האחרונה שלש עשרה תחלוק שלש עשרה על שלשה יעלה ארבעה תחברם עם האחד הנשאר מן החלוקה ויהיו חמשה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והיה זה כן לפי שהחמשית כאשר הוכתה על עצמה היתה תשיעית עוד הוכתה תשיעית על החמשית והיתה המעלה השלש עשרה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Apply this. | והקש על זה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Subtract the cube of the resulting root from the upper row and the remainder there is the first remainder. | גרע מעוקב השרש היוצא מהטור העליון והנשאר שם הוא הנשאר הראשון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Then take the extracted root, add it to one unit from the preceding rank and multiply it by the extracted root, multiply the result by three times the added unit and keep the result. | אח"כ קח השרש המוצא וחברהו עם אחד מהמעלה שלפניו וערוך זה על השרש המוצא והעולה תערוך על שלשה דמיוני האחד המוסף ושמור העולה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If the reserved is less than the first remainder, divide the first remainder by the reserved, but it is necessary that you make sure that you are left with the cube of the root resulting from the division, and that in the upper row remains also a number, whose ratio to the number you have divided is as the ratio of the resulting root minus one to the sum of the extracted root and the result. | ואם היה השמור פחות מהנשאר הראשון תחלק הנשאר הראשון על השמור אלא שצריך שתשמור שישאר לך מעוקב השרש היוצא בחלוק ושישאר גם כן בטור העליון מספר יהיה יחסו אל מה שחלקת כיחס השרש היוצא פחות אחד אל השרש המוצא עם העולה מקובצים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Meaning, if the root is 6 in a given rank and the resulting root is 5 of the preceding rank, there should remain a number from what you have divided, whose ratio to the divided number is approximately as the ratio of 4 to 65. | רצוני שאם היה השרש ו' ממעלה מונחת והיה השרש היוצא ה' ממעלה שלפניה הנה ראוי שישאר ממה שחלקת מספר יהיה יחסו אל המספר המחולק כיחס ד' אל ס"ה בקירוב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| That is very difficult for the first remainder. | וזה קשה מאד בנשאר הראשון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| However, from it on, it is enough that a little bit remains on the resulting root, since the ratio of the resulting root to the extracted root is small. | אמנם ממנו ולהלאה יספיק דבר מועט שישאר על מעוקב השרש היוצא למיעוט יחס שרש היוצא אל שרש המוצא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| And to make it easier for you, I give you a good reasonable way to follow regarding the first remainder. | ולהקל מעליך נתתי לך דרך טובה וקרובה תלך בה בנשאר הראשון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| It is this: investigate by how much the cube of the number that follows the extracted root exceeds the cube of the extracted root. | והיא זאת חקור כמה יוסיף מעוקב המספר הנמשך אל השרש המוצא לאחריו על מעוקב מספר השרש המוצא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Divide the first remainder by a tenth of this excess; the root resulting from the division are the units of rank that precedes the rank of the extracted root. | ועל עשירית היתרון תחלוק הנשאר הראשון והשרש היוצא מן החלוקה הם אחדים מהמעלה אשר לפני מעלת השרש המוצא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| After you complete this, be it in the first or the second way, multiply the extracted root by [the sum of] the extracted and the resulting roots, multiply the product by three times the resulting root and add the result to the cube of the resulting root, subtract the result from the first remainder and what remains is the second remainder. | ואחר שישלם לך זה אם בדרך הראשונה או בשנית תערוך השרש המוצא על השרש המוצא והיוצא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If the first remainder does not reach to the tenth part of the number by which we wanted to divide, do not conclude from this that it is impossible for you to place one in the rank that precedes the extracted root, but check if you add one to the extracted root in the rank that precedes it, then multiply the result by the extracted root, multiply the product by three times the addition, meaning the one added to the extracted root, and add the result to the cube of the addition: | ואם לא הגיע הנשאר הראשון לעשירית המספר אשר אמרנו לחלק עליו לא תשפוט מפני זה שלא יהיה אפשר שתשים בשרש המוצא אחד במעלה שלפניו אך תנסה אם תוסיף אחד על השרש המוצא במעלה שלפניה ותכה העולה על השרש המוצא ותערוך העולה מהכפל על שלשה דמיוני התוספת רצוני האחד המוסף על השרש המוצא ותחבר עם העולה מעוקב התוספת | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If it is not greater than the first remainder, subtract it from the first remainder and place one before the extracted root. | אם יהיה בלתי גדול מהנשאר הראשון תגרעהו מהנשאר הראשון ותשים אחד לפני השרש המוצא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If it is greater than the [first] remainder, it clear to you that there is nothing of this rank in this root, so you proceed with the one of the preceding rank that is third to the rank of the extracted root. | ואם היה גדול מהנשאר הנה נתבאר לך שאין בזה השרש דבר מהמעלה ההיא ותשוב לנהוג עם אחד מהמעלה האחרת אשר היא שלישית למעלת השרש המוצא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Meaning that you add it to the extracted root, multiply the sum by three times the addition that was added to the extracted root and divide the second remainder by the result, in a way that you are left with the cube of the result and even more, according to the mentioned ratio, but a small number that remains now is enough. | רצוני שתחברהו עם השרש המוצא והעולה תערוך על שלשה דמיוני התוספת שהוסף על השרש המוצא ועל העולה תחלוק הנשאר השני בדרך שישאר לך מעוקב העולה ויותר לפי היחס הנזכר אלא שמספר מעט שישאר עתה מספיק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The quotient that you have is the resulting root; write it in the row of the root in that rank. | והעולה בידך הוא השרש היוצא ותכתבהו בטור השרש במעלה ההיא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Multiply the extracted root by the sum of the extracted and resulting roots, then multiply the product by three times the resulting root, add the result to the cube of the resulting root and subtract the result from the remainder that you have. | ותערוך השרש המוצא על השרש המוצא והיוצא מקובצים והעולה תערוך על שלשה דמיוני השרש היוצא וחבר העולה עם מעוקב השרש היוצא והעולה תגרע מהנשאר אשר בידך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Like this you can further calculate more accurately until you reach to the [cube] root of the sought number. | וכזה תוכל לדקדק עד שתגיע אל שרש המספר המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| We want to give you examples of these ways one by one. | והנה נתן לך דמיונים לפי הדרכים האלה אחד אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה רצינו בזאת הצורה להוציא שרש א'ב'ג'ד'ה'ו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולפי שהמעלה האחרונה אינה מעוקבת הורדנוה אל שלפניה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה המעוקב הקרוב לזה המספר הוא תקי"ב ויסודו ח' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולפי שזאת המעלה היא רביעית שמנו השרש שהוא ח' בשנית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומעוקבם תקי"ב מהרביעית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
גרענום מתרנ"ד שיש לנו ברביעית ונשארו לנו קמ"ב ברביעית והוא הנשאר הראשון עם מה שנשאר בשאר המעלות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה לפי הדרך הראשון נערוך השרש המוצא שהוא ח' בשנית על ח' מהשנית א' מהראשונה ועלה ו' אלפים ת"פ | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ערכנו העולה על ג' דמיוני א' מהראשונה שהוא התוספת ועלה י"ט אלפים ות"מ | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקנו הנשאר הראשון על זה העולה ויעלה ז' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אלא שלא ישאר מהנשאר הראשון אחר שגרענו מהנשאר מעוקב מספר ז' מהראשונה אלא ה' אלפים וח' מאות וצ"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה יחסו אל הנשאר הראשון פחות מיחס ו' מהראשונה אל פ"ז מהראשונה שהוא השרש המוצא והיוצא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה שזה היחס הוא חלק מי"ד בקירוב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם נשים השרש היוצא מן החלוקה ו' יהיה הנשאר מספיק לזה היחס ר"ל ליחס ה' אל פ"ו שהוא חלק מי"ז בקירוב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
לפי שהנשאר הוא כ"ה אלפים תת"ן והוא מוסיף על חלק מי"ז במה שנשאר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולזה יהיה העולה ו' מהראשונה והוא השרש היוצא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונערוך ח' מהשנית שהוא השרש המוצא על השרש המוצא והיוצא ועלה ו' אלפים תת"פ | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ערכנום על ג' דמיוני ו' מהראשונה שהוא השרש היוצא ועלה קכ"ג אלפים תת"פ | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואולם מעוקב השרש היוצא הוא רי"ו חברנוהו עם העולה ועלה קכ"ד אלפים ונ"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
גרענום מהנשאר הראשון ונשאר י"ח אלפים רס"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונתברר לנו שלא נוכל להוסיף אחד על השרש לפי שהאחד המוסף על פ' הוסיף על המעוקב י"ט אלפים ותמ"א והם יותר ממה שישאר לנו עתה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם רצית להקל מעליך אחר שחלקת על י"ט אלפים ות"מ ושמת העולה ו' ונשאר לך כ"ה אלפים תס"ה אחר גרעון מעוקב מהנשאר תערוך השרש המוצא על השטח ההוה מהשרש היוצא פחות אחד על שלשה דמיוני השרש המוצא והעולה תגרע מכ"ה אלפים תס"ה והוא המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
משל זה שתערוך פ' על שטח ה' בי"ח ויעלה ז' אלפים ומאתים גרעם מכ"ה אלפים תס"ה וישאר לך י"ח אלפים רס"ה וזה מסכים למה שנשאר קודם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ובזה התבאר לך הסבה במה שצויתיך להשמר שיהיה לך היחס הנזכר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והיה זה כן לפי שהנערך מפ' אל פ"א הוכה בג' והעולה בו' וזה שוה לשטח פ"א בשטח פ' בי"ח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכבר היה ראוי שיוכה לפי מה שקדם שטח פ"ו בשטח פ' בי"ח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והוא מבואר שאם נחבר עם שטח פ"א בשטח פ' בי"ח שטח ה' בשטח פ' בי"ח יהיה העולה שוה לשטח ההוה ממספר פ"ו בשטח פ' בי"ח אשר הוא המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Apply this. | והקש על זה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם נהגנו בזה הדרך השנית והוא היותר קלה על צד הקירוב נחלק הנשאר שהוא קמ"ב אלפים שכ"א על עשירית יתרון מעוקב ט' מהשנית שהוא המספר הנמשך אל השרש המוצא לאחריו על מעוקב ח' מהשנית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה היתרון הוא רי"ז אלף ועשיריתו הוא רי"ז עשיריות והם כמו כ"א אלפים וז' מאות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקנו הנשאר הראשון על כ"א אלפים וז' מאות ועלה ו' שלמים והוא השרש היוצא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ערכנו שרש המוצא שהוא פ' על השרש המוצא והיוצא ועלה ו' אלפים תת"פ | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ערכנום על שלשה דמיוני השרש היוצא ועלה קכ"ג אלפים תת"מ | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חברנו עם זה העולה מעוקב היוצא שהוא רי"ו ועלה קכ"ד אלפים ונ"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
גרענום מן הטור העליון ונשאר י"ח אלפים רס"ה והוא הנשאר השני | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Apply this. | והקש על זה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואמנם בזה הנשאר השני תנסה אם תוסיף ראשון אחד שהוא המדרגה הנמשכת לפני השרש על השרש המוצא שהוא פ"ו כמה יתוסף המעוקב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ושמור העולה וחלק הנשאר השני על העולה והשמר שישאר לך מעוקב השרש היוצא ושטח השרש היוצא פחות אחד בשטח השרש המוצא בשלשה דמיוני העולה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ערכנו פ"ו שהוא השרש היוצא על פ"ו וראשון אחד וערכנו העולה על ג' ראשונים ועלה שס"ט שלמים נ"ב ראשונים ג' שניים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקנו הנשאר השני על העולה ועלה מ"ט והם ראשונים אלא שלא ישאר מהנשאר השני כמו יחס מ"ח ראשונים אל פ"ו ומ"ט ראשונים שהוא חלק מק"ט בקירוב לפי [ש]הנשאר הוא כמו ק"מ שלמים בקרוב והוא מהנשאר פחות מחלק מק"ט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולזה לא נכתוב בטור השרש כי אם מ"ח ראשונים כי אז יספיק לנו הנשאר אל זה היחס | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ערכנו פ"ו אל פ"ו מ"ח ראשונים והעולה על שלשת דמיוני מ"ח ראשונים ועלה י"ז אלפים תתקט"ו שלמים ול"א ראשונים וי"ב שניים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חברנו עם זה מעוקב מ"ח ראשונים שהוא השרש היוצא והוא ל' ראשונים מ"ג שניים י"ב שלישיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
גרענו העולה מהשאר השני ונשאר שנ"ד שלמים נ"ח ראשונים ד' שניים מ"ח שלישיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה ננסה אם נוסיף שני אחד על השרש המוצא כמה יתוסף המעוקב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ערכנו פ"ו שלמים מ"ח ראשונים ושני אחד וערכנו העולה על ג׳ שניים ועלה בקירוב ו' שלמים י"ז ראשונים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ושמרהו כי לא תצטרך לנסיון אחר מכאן והלאה וזה למיעוט יחס השרש היוצא אצל השרש המוצא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקנו הנשאר על זה השמור ועלו נ"ה והם שניים והעולה על שלשה דמיוני נ"ה שניים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועם העולה חברנו מעוקב נ"ה שניים שהוא השרש היוצא ועלה שמ"ה שלמים כ"ב ראשונים מ"ח כ"ט י"ו י"ב נ"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
גרענוהו מהנשאר ונשאר ג' שלמים ל"ה ראשונים י"ו י"ח מ"ג מ"ז ה' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקנו על חלק מהששים מהשמור שהוא ו' ראשונים י"ז שניים והוא השמור השני ועלה ל"ד והם שלישיים כי השלישי הוא החלק מהששים מהם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכבר הוסיף השני המעוקב ו' שלמים י"ז ראשונים בקירוב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ערכנו השרש המוצא שהוא פ"ו שלמים מ"ח ראשונים נ"ה שניים על פ"ו שלמים מ"ח ראשונים נ"ה שניים ל"ד שלישיים והעולה ערכנו על שלשת דמיוני השרש היוצא שהוא ל"ד שלישיים וחברנו עם העולה מעוקב ל"ד שלישיים ועלה ג' שלמים ל"ג ראשונים ל"ב מ"ד כ"ה כ"ז ל"ז מ"ז נ"ה ד' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
גרענום מהנשאר ונשאר ראשון אחד מ"ג ל"ד י"ח כ"ט כ"ח נ"ו ד' נ"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקנוהו על חלק מהששים מהשמור השני שהוא ו' שניים י"ז שלישיים והוא השמור השלישי ועלה י"ו והם רביעיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואין צריך לדקדק עוד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תרצה תוכל לדקדק בזה הדרך כפי מה שתרצה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה שרש זה המספר הדרוש העקובי הוא פ"ו שלמים מ"ח ראשונים נ"ה שניים ל"ד שלישיים י"ו רביעיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| You shoud know that in the second way, which I gave you for the first remainder, if there are fractions in the rank that precedes the extracted root, you should divide by sixty the excess of the cube of the number that follows the exctracted root over the cube of the extracted root, which is obvious by itself. | וראוי שתדע כי בדרך השנית שנתתי לך בנשאר הראשון אם היתה המדרגה שלפני שרש המוצא שברים ראוי שתחלק על ששים יתרון מעוקב המספר הנמשך אל השרש המוצא לאחריו על מעוקב השרש המוצא וזה מבואר בעצמו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Another way to extract the cube root of a given number when there are integers in its root: | דרך אחרת להוציא שרש מספר מונח העקוביי אשר יהיו ביסודו שלמים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Know that it is very easy to extract the cube root of the number that is smaller than one thousand, compared to what is greater than it. | דע כי המספר שלא יגיע לאלף יקל מאד להוציא שרשו העקוביי בערך אל מה שלמעלה ממנו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| I will to instruct you how to lower a great number to a smaller number, by explaining that the multiplication of a given number by 3 seconds and 36 thirds is equal to dividing it by one thousand. | ואודיעך איך תוריד מספר רב אל מספר מעט אחר שאבאר שהכאת מספר מונח על ג' שניים ל"ו שלישיים שוה לחלוקו על אלף | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Because a thousand times 3 seconds and 36 thirds is one integer. | וזה שאלף פעמים ג' שניים ל"ו שלישיים יהיה אחד שלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Therefore, 3 seconds and 36 thirds are a thousandth part of one integer. | אם כן ג' שניים ל"ו שלישיים הם חלק מאלף באחד שלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| When this is established, you should divide the number by one thousand and multiply the remainder that cannot be divided by 3 seconds and 36 thirds; this is the first reduction. | וכאשר התישב זה הנה ראוי שתחלוק המספר על אלף והנשאר שלא יבוא לחלוק תכפלהו על ג' שניים ל"ו שלישיים והיא ההורדה הראשונה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If the result is not less than one thousand, divide it again by one thousand in the previous way; this is the second reduction. | ואם העולה בלתי קטן מאלף תשוב לחלקו על אלף בדרך הקודמת והיא ההורדה השנית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| And so you do not stop reducing until the number is smaller than one thousand and you count the number of reductions. | וכן לא תסור להוריד עד שיהיה המספר קטן מאלף ותמנה מספר ההורדות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| You extract the root of the small number and calculate very precisely until the sixths, or as much as you wish, because in this way it is very easy. | ותוציא שרש המספר הקטן ותדקדק עד ששיים או כפי מה שתרצה כי יקל מאד בזה הדרך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| You multiply the result by the number that consists of as many tens as the number of reductions. | והעולה בידך תערכהו על המספר המורכב ממספרי עשרה כמספר ההורדות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| This number is equal to the unit of the rank whose position exceeds the number of reductions by one. | וזה מספרם שוה לאחד מהמעלה אשר מספר גבהם מוסף אחד על מספר ההורדות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Then, you have the sought. | ויצא לך המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה אם רצית להוציא שרש א'ב'ג'ד'ה'ו'ז'ח'ט'ה' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תורידהו הנה שלשה הורדות כדי שיהיה המספר העולה בידך באחרונה פחות מאלף | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה המספר העולה בידך בהורדה באחרונה ה' שלמים נ"ט ראשונים ט"ו ל"ג ז' נ"ד ל"ג 0 ל"ד ל"ו תשיעיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה המעוקב הקרוב למה שבמדרגה האחרונה שהיא מעוקבת היא אחד ושרשו אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותכתוב אחד בטור השרש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
גרעת מעוקבו מהטור העליון ונשארו ד' שלמים נ"ט ראשונים ומה שנמשך לזה מהשברים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה ידעת שמעוקב שנים יוסיף על מעוקב אחד ז' שלמים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה המדרגה שלפני השרש המוצא היא ממדרגת השברים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וחלקת הנשאר בטור העליון על חלק מששים מז' שלמים שהוא ז' שברים ראשונים ועלה מ"ב והם חלקים מששים באחד שלם ולזה יהיו שברים ראשונים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ערכת השרש המוצא שהוא אחד על אחד ומ"ב ראשונים וערכת העולה על שלשת דמיוני השרש היוצא שהוא מ"ב ראשונים וחברנו עם העולה מעוקב מ"ב ראשונים שהוא השרש היוצא ועלה ג' שלמים מ"ב ראשונים ח' שלישיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
גרענום מהנשאר ונשאר אחד שלם י"ז ראשונים ט"ו כ"ה ז' נ"ד ל"ד ל"ג ל"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה ראוי שננסה מה יוסיף ראשון אחד על המעוקב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ערכנו השרש המוצא שהוא אחד שלם ומ"ב ראשונים על אחד ומ"ג ראשונים וערכנו העולה על ג' דמיוני התוספת שהוא ראשון אחד ועלה בקירוב ח' ראשונים מ"ו שניים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקת עליהם הנשאר ועלה ח' והם ראשונים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הוספת על השרש המוצא ח' ראשונים וערכת א' מ"ב ראשונים על א' ונ' ראשונים והעולה על כ"ד ראשונים שהוא שלשה דמיוני השרש היוצא שהוא ח' ראשונים ועלה אחד שלם י"ד ראשונים נ"ו ל"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
גרענום מהנשאר ונשארו ב' ראשונים י"ח שניים נ"ג ז' נ"ד ל"ד ל"ג ל"ו והוא הנשאר פחות ממה שיוסיף ראשון אחד על המעוקב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה ראוי שננסה מה יוסיף שני אחד על המעוקב הזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ערכנו השרש המוצא שהוא א' ונ' ראשונים על א' ונ' ראשונים ושני אחד והעולה על שלשה דמיוני התוספת שהוא א' שניים ועלה י' שניים מ"ו שלישיים בקרוב והוא השמור הראשון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקת עליו הנשאר ועלה י"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ערכת אחד ונ' ראשונים על אחד ונ' ראשונים י"ג שניים והעולה על שלשה דמיוני השרש היוצא שהוא י"ג שניים וחברת העולה עם מעוקב י"ג שניים ועלה ב' ראשונים י"א כ' ל"ו ל"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
גרענום מהנשאר ונשאר ז' שניים ל"ב ל"ז מ"ח מ"ז כ"ג ל"ד ל"ג ל"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקנו זה הנשאר על חלק מס' מזה השמור הראשון שהוא י' שלישיים ו' רביעיים והוא השמור השני ועלה מ"ד והם שלישיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ערכת אחד ונ' ראשונים י"ג שניים על אחד ונ' ראשונים י"ג שניים מ"ד שלישיים והעולה על שלשה דמיוני השרש היוצא שהוא מ"ד שלישיים וחברת העולה עם מעוקב מ"ד שלישיים ועלה ז' שניים כ"ה שלישיים כ"ו נ"ח א' נ' נ"ג מ"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
גרענום מהנשאר ונשאר ז' שלישיים ט' נ"א כ"ב כ"ד נ"ט נ"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקנו הנשאר על חלק מששים מהשמור השני שהוא י' רביעיים וז' חמשיים בקרוב והוא השמור השלישי ועלה מ"ב והם רביעיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ערכת אחד ונ' ראשונים י"ג שניים מ"ד שלישיים על אחד ונ' ראשונים י"ג שניים מ"ד שלישיים מ"ב רביעיים והעולה על שלשה דמיוני השרש היוצא שהוא מ"ב רביעיים וחברת העולה עם מעוקב מ"ב רביעיים ועלה ז' שלישיים ה' ט"ו כ"ד י"ו כ"א י"ג כ"ב מ"ח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
גרענום מהנשאר ונשאר ד' רביעיים ל"ה חמשיים כ"ח י"ח כ"ג ל"ד מ"ו ל"ז י"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואין צורך לדקדק עוד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תרצה עתה תוכל לדקדק לשני מעלות יחד ותהיה קרוב מאד אל המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה שאתה אם חלקת הנשאר על חלק מהששי מהשמור השלישי שהוא י' חמשיים י"ו ששיים ועלה כ"ז והם חמשיים וי"ו והם ששיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולו תחקור תמצא שלא יגיע הקרוב לב' שלישיות חמשית אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולזה יהיה שרש זה המספר המורד העקוביי אחד ונ' ראשונים י"ב שניים מ"ד מ"ב כ"ו י"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ערכנו זה השרש על אלף לפי שההורדות היו ג' ועלה אלף תתל"ז ט' ראשונים ה' שניים ז' שלישיים ל"ד רביעיים מ' חמשיים מ' ששיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והתבאר בכמו זה הביאור הקודם במספר המורד להוציא שרשו הרבועיי שזהו השרש העקוביי למספר הרב בקרוב ושיחס הקרוב אל הקרוב כיחס המספר אל המספר ר"ל אלף אלפי אלפים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה שהמספר הגדול ימנה הקטן כמספר אחדי אלף אלפי אלפים לפי שיחס המעוקב אל המעוקב הוא יחס צלעו אל צלעו משולש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר תנהיג זה יתבאר לך בכמו הבאור הקודם שיחס הקירוב אשר ממעוקב השרש הגדול אצל מספר הגדול אל הקרוב אשר ממעוקב השרש הקטן אצל המספר הקטן הוא גם כן אלף אלפי אלפים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The way of extracting the [cube] root of a given cubic number that does not contain only integers when the fractions are not sexagesimal fractions. | דרך להוציא שרש מספר מעוקב מונח בלתי מקיף בשלמים העקוביי והשברים אינם משברי חכמי התכונה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Take the denominator of all fractions: if the denominator is not a cubic number, multiply the number by the cube of the denominator or by the denominator if it is a cubic number, then divide the result by the cubic root, by which you have multiplied the number, and it is the sought. | קח המורה הראשון לכל השברים ואם לא היה המורה מעוקב ערוך המספר על מעוקב המורה או על המורה אם היה מעוקב והעולה חלק על שרש העקוביי שערכת עליו המספר והוא המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה אם רצית לדעת שרש מ"ד שלמים וה' שביעיות רביעית וי"ג שביעיות שביעית רביעית רביעית וכ"ז שביעיות שביעית שביעית רביעית רביעית רביעית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
לקחנו המורה הראשון לאלו השברים ושברי השברים בכלל והיה המספר המורכב ממספרי ז'ז'ז'ד'ד'ד' וזה שוה להכאת מעוקב ז' במעוקב ד' אם כן המורה לאלה השברים הוא מעוקב ושרשו העקובי' הוא שטח ז' בד' שהוא כ"ח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ערכנו מ"ד עם השברים על המורה והוצאנו שרש העולה והנה צ"ט חלקנו צ"ט על כ"ח ועלה ג׳ שלמים וט"ו חלקים מכ"ח באחד וככה המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The way of extracting cubic root of sexagesimal fractions | דרך הוצאת השרשים העקוביים משברים מונחים משברי התכונה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| First, check if the last rank of the row is a cubic one; if it is not cubic, lower the rank to the preceding [rank] until it reaches to a cubic rank. | חקור תחלה על המדרגה האחרונה שבטור אם היא מעוקבת ואם אינה מעוקבת הורד המדרגה אל שלפניה עד שתגיע למדרגה מעוקבת | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Then, extract the approximated small cubic root of the number you find in that rank. | והמספר שתמצא במדרגה ההיא תוציא שרשו העקוביי הקרוב ואולם המעט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Place the result in the rank that I will explain: divide the position of the rank by 3 and place the result there. | ותשים העולה במעלה אשר אבארה והוא שתחלק שפלות המעלה על ג' ושם תשים העולה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
משל זה אם היתה המדרגה מדרגת הששיים הנה כאשר תחלק שפלות המעלה שהוא ו' על ג' יעלה ב' ולזה תשים העולה במדרגה השנית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה שהשניים הוכו בעצמם והיו רביעיים והרביעיים הוכו בשניים והיו ששיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Then, you cube of the extracted root and subtract it from the upper row. | ותוציא מעוקב השרש המוצא ותגרעהו מהטור העליון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| You examine the remainder: if you add one in the rank that precedes the extracted root, by how much will the cube increase? | והנשאר לך תנסה אם תוסיף אחד במדרגה שלפני השרש המוצא כמה יתוסף המעוקב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Divide the remainder by the result, in a way that you are left with the cube of the resulting root and its ratio to the divided number is the same as the ratio of the resulting root minus one to [the sum of] the extracted and resulting roots. | ועל העולה חלק הנשאר בדרך שישאר לך מעוקב השרש היוצא ומספר יחסו אל המספר המחולק כיחס השרש היוצא פחות אחד אל השרש המוצא והיוצא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Finally, you complete its extraction according to the previous rule. | וסוף דבר תנהיג בשלמות הוצאתו כמנהג הקודם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה אם רצית לדעת שרש נ"ט ראשונים כ"ג שניים ז' שלישיים מ' רביעיים העקוביי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הורד הראשונים והשניים אל מדרגת השלישיים ועלה שם ז'ח'ז'ג'א'ב' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הוצאנו השרש הקרוב לזה המספר והוא נ"ט והם ראשונים לפי מה שקדם והנשאר הוא ח' אלפים ת"ח שלישיים מ' רביעיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה ראוי שננסה מה יוסיף שני אחד על המעוקב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ערכנו נ"ט ראשונים על נ"ט ראשונים ושני אחד והעולה על ג' שניים ועלה ב' שניים נ"ה שלישיים בקרוב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקנו עליו הנשאר והנה השרש היוצא מן החלוקה הוא מ"ז והם שניים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונשאר הראוי לפי יחס מ"ו שניים אל נ"ט ראשונים מ"ז שניים שהוא חלק מע"ח בקרוב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ערכנו נ"ט ראשונים על נ"ט ראשונים מ"ז שניים והעולה על ג' דמיוני מ"ז שניים וחברנו עם העולה מעוקב מ"ז שניים ועלה ב' ראשונים י"ח שניים נ"ו כ"ו כ"ג כ"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
גרענום מהנשאר ונשארו קי"ט שלישיים י"ג רביעיים ל"ו ל"ח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וראוי שננסה מה יוסיף שלישי אחד על המעוקב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולא נצטרך עוד לבחינה למיעוט היחס אצל השרש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה עלה ב' שלישיים נ"ט רביעיים בקרוב והוא השמור הראשון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה השרש היוצא הוא מ' והם שלישיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ערכנו נ"ט ראשונים מ"ז שניים על נ"ט ראשונים מ"ז מ' והעולה על שלשה דמיוני מ' שלישיים וחברנו עם העולה מעוקב מ' שלישיים ועלה קי"ט שלישיים ט' רביעיים כ"ה ב' נ"ז מ"ו מ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
גרענוהו מהנשאר ונשאר ד' רביעיים י"א כ' י"ג כ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואין צריך לדקדק עוד ואם תרצה תוכל לדקדק שתי מעלות יחד וזה שתחלק הנשאר על חלק מששים מהשמור ויעלה בידך רביעי אחד וכ"ה חמשיים והנה השרש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם כן הוא נ"ט ראשונים מ"ז שניים מ' שלישיים א' רביעי כ"ה חמשיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Apply this. | והקש על זה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The way of extracting a mean number between two different proportional numbers | דרך הוצאת מספר אמצעי ביחס בין שני מספרים מונחים מתחלפים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Multiply the one by the other and extract the square root of the product; the result is the required. | ערוך האחד על האחר והוצא שרש העולה הרבועי והוא המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה אם רצית שתמצא המספר האמצעי ביחס בין שני מספרי ד' וי"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ערוך ד' על י"א והנה מ"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הוצא את יסודו הרבועי ועלה ו' שלמים ל"ז ראשונים נ"ט שניים מ"ב שלישיים והוא אמצעי ביחס בין מספרי ד' וי"א בקירוב גדול | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The way of extracting two proportional numbers that are mean between two given different numbers. | דרך הוצאת שני מספרים אמצעים ביחס בין שני מספרים מונחים מתחלפים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Divide the greater by the smaller, take the cube root of the quotient and this is the first reserved. | חלק הגדול על הקטן והעולה בחלוק הוצא את יסודו העקוביי והוא השמור הראשון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Take the square of the cube root and this is the second reserved. | גם הוצא מרובע יסודו העקוביי והוא השמור השני | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Multiply the first reserved by the smaller number of the two given numbers and you will get the number that succeeding the smaller number in the required ratio. | ערוך השמור הראשון על המספר הקטן משני המספרים המונחים ויצא לך המספר הנמשך ביחס הדרוש למספר הקטן | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Multiply the second reserved by the smaller number and you will get the number that is third to the smaller number in the required ratio. This is the sought. | ערוך השמור השני על המספר הקטן ויצא לך המספר השלישי ביחס הדרוש למספר הקטן והנה המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה אם רצית שנמצא שני מספרים אמצעים ביחס בין ט"ו וכ"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקנו כ"ה על ט"ו ועלה אחד שלם ומ' ראשונים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הוצאנו יסודו העקובי ועלה אחד שלם י"א ראשונים ח' שניים ט' שלישיים י"ט רביעיים ד' חמשיים ל' ששיים והוא השמור הראשון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה מרובע השמור הראשון הוא אחד כ"ד ראשונים כ' ל"ה מ"ה ל"ד י' כ"ח כ"ז נ"א כ' ט"ו והוא השמור השני | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ערכנו השמור הראשון על המספר הקטן שהוא ט"ו ועלה י"ז שלמים מ"ז ראשונים כ"ד מ"ו ז' ל' וזה המספר הוא השני למספר ט"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ערכנו השמור השני על ט"ו ועלה כ"א שלמים ה' ח' נ"ו כ"ג ל"ב ל"ז ו' נ"ז נ"ג מ"ה והוא המספר השלישי למספר ט"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואומר שאלו שני המספרים הם אמצעים ביחס הקירוב בין ט"ו ובין כ"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה שכל מעוקב כבר יפול בינו ובין האחד שני מספרים אמצעים והאחד מהם הוא שרש המעוקב והאחר מרובעו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה שיחס האחד אל שרש המעוקב כיחס השרש אל המרובע וכיחס המרובע אל המעוקב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כבר לוקחו לאלו הארבעה כפלים שוים והוא ט"ו וזה שהאחד הוכה בט"ו והיה ט"ו והמעוקב הוכה בט"ו והיה כ"ה והמספרים האמצעים הוכו בט"ו גם כן | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה א"כ אלו הארבעה הכפולים בט"ו הם גם כן מתיחסים בכמו היחס הקודם בעינו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Apply this. | והקש על זה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Chapter Six – Ratios |
השער הששי בערכים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| והוא הקש המספרים קצתם אל קצת | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| You already know that for every four proportional numbers, the product of the first by the fourth is as the product of the second by the third. | כבר ידעת שכל ארבעה מספרים מתיחסים הנה שטח הראשון ברביעי כמו שטח השני בשלישי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Since it is so, we shall explain to you, if there are any given numbers and we have a second given number that corresponds to one of these numbers, how you may find the other corresponding numbers, so that the corresponding numbers are in the former ratio. | וכאשר היה זה כן הנה נבאר לך אם היו מספרים מה מונחים והיה לנו מספר אחד מונח שני והוא גיל אחד מונח מהמספרים ההם איך תוציא שאר המספרים הגיליים עד שיהיו המספרים הגיליים בכמו זה היחס הקודם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| You should know that if you multiply one of the numbers by the given second number and divide by its corresponding number, you will get the corresponding number of the number that was multiplied by the given second number. | ראוי שתדע שאם תכה אחד מהמספרים במספר המונח השני ותחלוק על גילו יצא לך המספר הגיליי למספר אשר הוכה על המספר המונח השני | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה שהמספרים המונחים מספר א'ב'ג'ד'ה' והיה מספר ז' גיליי למספר ד' ונרצה שנמצא המספרים הגיליים למספרי א'ב'ג'ד'ה' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה נכה ז' בא' ונחלק על ד' ויצא לנו כ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה מפני ששטח א' בז' כמו שטח כ' בד' הנה יחס א' אל ד' כיחס כ' אל ז' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועל התמורה הנה יהיה יחס א' אל כ' כיחס ד' אל ז' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ובזה התבאר שאם הוכה ב' בז' וחולק על ד' ויהיה העולה ט' שמספר ט' הוא הגיליי למספר ב' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וגם כן כבר יוכה ג' בז' ויחולק על ד' ויצא ח' הנה ח' גיליי למספר ג' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וג"כ כבר יוכה ה' בז' ויחולק על ד' ויצא ל' הנה ל' גיליי לה' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה כבר מצאנו המספרים הגיליים לא'ב'ג'ה' והם כ'ט'ח'ז'ל' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והוא מבואר שמספרי כ'ט'ח'ז'ל' על יחס מספרי א'ב'ג'ד'ה' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומש"ל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Even if we do not know the number of the series of the corresponding terms, but we know the sum of two or three of the corresponding numbers, it is possible for us to find from this the [corresponding] numbers. | וגם כן אם לא היה נודע לנו מספר מהמספרים הגיליים ונדע לנו מקובץ שניים מהגיליים או שלשה מהם הנה כבר אפשר שנעמוד מזה על המספרים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והמשל שיהיה נודע לנו במשלנו זה שמקובץ כ'ז'ל' כמו מספר מ' ורצינו לעמוד מזה על המספרים הגיליים למספרי א'ב'ג'ד'ה' המונחים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה נשים מקובץ גילי מספרי כ'ז'ל' והם מספרי א'ד'ה' מספר נ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכמו יחס נ' אל מ' כן נשים יחס כל מספרי א'ב'ג'ד'ה' אל גילו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולזה הנה נכה מ' בא' ונחלק על נ' ויצא מספר כ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ובזה הדרך נוציא מספרי כ'ט'ח'ז'ל' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונאמר שמספרי כ'ט'ח'ז'ל' הם המספרים המבוקשים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
המופת שיחס נ' אל מ' כיחס א' אל כ' וכיחס ד' אל ז' וכיחס ה' אל ל' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר קבצנו הנה יחס נ' אל מ' כיחס מספרי א'ד'ה' מקובצים אל מספרי כ'ז'ל' מקובצים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר המירונו הנה יחס נ' אל א'ד'ה' מקובצים כיחס מ' אל כ'ז'ל' מקובצים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אבל נ' שוה למספרי א'ד'ה' מקובצים אם כן מ' שוה למספרי כ'ז'ל' מקובצים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם כן כבר מצאנו המספרים הגיליים למספרי א'ב'ג'ד'ה' ומספרי כ'ז'ל' מהם מקובצים שוים למספר מ' המונח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Even if it is only known for us concerning the corresponding numbers that the excess of a number of them, whose corresponding position is known, or of a sum of numbers, whose corresponding positions are known, over a number of them, whose corresponding position is known, or over a sum of known numbers, is a given number, it is possible for us to find the corresponding numbers each in its position. | וג"כ אם לא יודע לנו מהגיליים אלא שיתרון מספר מהם ידוע הגיליות או מקובץ מספרים ידועי הגיליות על מספר מהם ידוע הגיליות או מקובץ מספרים ידועים הוא מספר מונח הנה אפשר לנו שנעמוד מזה על המספרים הגיליים איש על מקומו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והמשל במשלנו זה הקודם שיודע לנו שמקובץ מספרי ב'ח' מוסיף על מספר ל' מספר מ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם רצינו לעמוד מזה על ידיעת המספרים הגיליים למספרי א'ב'ג'ד'ה' הנה נשים מקובץ א'ג' מספר נ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונבאר שמספר נ' הוא מוסיף על מספר ה' וזה כי לפי שהיה יחס א' הידוע אל כ' הנעלם הוא יחס ג' הידוע אל ח' הנעלם והוא יחס ה' הידוע אל ל' הנעלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה אם כן יחס א'ג' מקובצים אל ה' כיחס כ'ח' מקובצים אל ל' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אבל מקובץ כ'ח' מוסיף על ל' אם כן מקובץ א'ג' מוסיף על ה' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונשים יתרון נ' על מספר ה' מספר ס' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה נכה א' במ' ונחלק על ס' ויצא לנו מספר כ' והוא גיליי למספר א' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן לא נסור עד שיצאו לנו מספרי כ'ט'ח'ז'ל' ונבאר שמספרי כ'ט'ח'ז'ל' הם המספרים המבוקשים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
המופת שיחס ס' אל מ' הוא יחס כל מספר ממספרי א'ב'ג'ד'ה' אל גילו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם כן יחס ט' אל מ' כיחס מקובץ א'ג' אל מקובץ כ'ח' וכיחס ה' אל ל' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר הבדלנו הנה יחס ס' אל ה' כיחס יתרון כ'ח' מקובצים על ל' אל ל' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר המירונו הנה יחס ס' ליתרון כ'ח' על ל' כיחס ה' אל ל' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר היה גם כן יחס ט' אל מ' כיחס ה' אל ל' א"כ יתרון כ'ח' על ל' הוא מ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
א"כ מספרי כ'ט'ח'ז'ל' הם גיליים למספרי א'ב'ג'ד'ה' ויתרון כ'ח' מקובץ על ל' הוא מ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והוא מש"ל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Even if we only know of the corresponding unknown numbers that a number of them, whose corresponding position is known, or a sum of numbers of them, whose corresponding positions are known, exceed over a part or parts of numbers of them, whose corresponding positions are known, by a given number, it is already possible for us to find the corresponding numbers for the given numbers. | וגם כן אם לא היה נודע מהמספרים הגיליים הנעלמים אלא שמספר מהם ידוע הגיליות או מקובץ מספרים מהם ידועי הגיליות מוסיף על חלק או חלקים ממספרים מהם ידועי הגיליות מספר מונח הנה כבר אפשר לנו שנעמוד מזה על ידיעת המספרים הגיליים למספרים המונחים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| In our example it is known for us that T exceeds over certain given parts of the sum of K, C by M. | ויהיה נודע לנו במשלנו זה שמספר ט' מוסיף על חלקים מונחים ממספרי כ'ז' מקובצים מספר מ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| We define these parts of the sum of A, D as the number N. | הנה נשים החלקים ההם ממספרי א'ד' מקובצים מספר נ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| We explain that B is greater than N.
|
ונבאר שמספר ב' מוסיף על מספר נ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| This is because the ratio of the known B to the unknown T is as the ratio of the known A to the unknown K.
|
וזה שיחס ב' הידוע אל ט' הנעלם הוא כמו יחס א' הידוע אל כ' הנעלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Hence, the ratio of B to T is as the ratio of the sum of A, D to the sum of K, Z.
|
אם כן יחס ב' אל ט' כיחס מקובץ א'ד' אל מקובץ כ'ז' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| But, the ratio of the sum of A, D to the sum of K, Z is as the ratio of N to the given parts of K, Z. | אבל יחס מקובץ א'ד' אל מקובץ כ'ז' הוא כיחס נ' אל החלקים המונחים ההם ממספרי כ'ז' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| It is clear that the ratio of A, D to N is as the ratio of K, Z to these given parts of K, Z. | וזה הוא מבואר שיחס א'ד' אל נ' הוא כיחס כ'ז' אל החלקים ההם המונחים ממספרי כ'ז' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If we switch the terms, the assertion is verified. | וכאשר המירונו התאמת המאמר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| So, the ratio of B to T is as the ratio of N to the given parts of K, Z. | הנה אם כן יחס ב' אל ט' הוא כמו יחס נ' אל החלקים המונחים ממספרי כ'ז' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| When we switch the terms, then the ratio of B to N is as the ratio of T to the given parts of the numbers K, Z. | וכאשר המירונו הנה יחס ב' אל נ' הוא כיחס ט' אל החלקים המונחים ממספרי כ'ז' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| But, T is greater than these parts, so B is greater than N.
|
אבל מספר ט' מוסיף על החלקים הנה אם כן מספר ב' מוסיף על נ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| We define its excess over N as the number E.
|
ונשים יתרונו על נ' מספר ע' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| We multiply A by M and divide by E; the result is K that corresponds to A.
|
הנה נכה א' במ' ונחלק על ע' ויצא כ' והוא גיליי למספר א' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| So, we do not stop until we find the numbers K, T, C, Z, L and we say that K, T, C, Z, L are the sought numbers. | וכן לא נסור עד שיצאו לנו מספרי כ'ט'ח'ז'ל' ונאמר שמספר כ'ט'ח'ז'ל' הם המספרים המבוקשים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Proof: the ratio of E to M is as the ratio of any of the numbers A, B, G, D, H to its corresponding. | המופת שיחס ע' אל מ' הוא יחס כל מספר ממספרי א'ב'ג'ד'ה' אל גילו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| So the ratio of E to M is as the ratio of the sum of A, D to the sum of K, Z and as the ratio of B to T.
|
אם כן יחס ע' אל מ' כיחס מקובץ א'ד' אל מקובץ כ'ז' וכיחס ב' אל ט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Hence, since the ratio of N to A, D is as the ratio of E to K, Z, the ratio of N to E is as the ratio of A, D to K, Z by switching the terms.
|
הנה מפני שיחס נ' אל א'ד' כיחס ע' אל כ'ז' יהיה יחס נ' על ע' כיחס א'ד' אל כ'ז' על התמורה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| But, the ratio of A, D to K, Z is as the ratio of B to T.
|
אבל יחס א'ד' אל כ'ז' הוא כיחס ב' אל ט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| So, the ratio of N to E is as the ratio of B to T.
|
אם כן יחס נ' אל ע' הוא כיחס ב' אל ט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If we switch and reverse, the ratio of B to N is as the ratio of T to E.
|
וכאשר המירונו והפכנו הנה יחס ב' אל נ' כיחס ט' אל ע' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| But, B exceeds over N by the number S.
|
אבל ב' מוסיף על נ' מספר ס' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| So, T must be larger than E.
|
הנה אם כן ט' מוסיף על ע' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The ratio of B to S is as the ratio of T to M by switching [the inner terms].
|
וכבר היה יחס ב' אל ס' כיחס ט' אל מ' על התמורה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Hence, the excess of T over E is M.
|
אם כן יתרון ט' על ע' הוא מ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| So, the numbers K, T, C, Z, L are the corresponding to the numbers A, B, G, D, H, and the excess of T over the given parts of K, Z is M. | אם כן מספרי כ'ט'ח'ז'ל' הם הגיליים למספרי א'ב'ג'ד'ה' ויתרון מספר ט' על החלקים המונחים ממספרי כ'ז' הוא מ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Q.E.D. | והוא מה ש"ל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| זה הוא מה שרצינו להציע והוא מועיל מאד בזה השער | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The author writes: The sixth chapter of this section is complete and with it this entire book is complete. | כתב המחבר נשלם השער הששי מזה המאמר ובהשלמו נשלם זה הספר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Glory to God alone! | והתהלה לאל לבדו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| It was completed at the beginning of Nissan at the eighty-first year of the sixth millennium when I had reached the thirty-third year of my life. | והיתה השלמתו בראש ניסן של שנת שמונים ואחת לפרט האלף הששי בהגיעי לשנת שלשים ולשלש משנותי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Praise be to the Helper! | וברוך העוזר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Word Problems |
|||||||||||||||||||||||||||||||||||||||||||||||||
| We present to you various problems, so that you may understand from them everything that is similar to them. | והנה [718]נסדר לך שאלות מתחלפות עד שתבין מהם כל מה שידמה להם | ||||||||||||||||||||||||||||||||||||||||||||||||
Find a Number Problems |
|||||||||||||||||||||||||||||||||||||||||||||||||
|
שאל שואל לקחנו חלק מונח או חלקים מונחים ממספר נעלם והיה מספר מונח כמה מספר הנעלם | ||||||||||||||||||||||||||||||||||||||||||||||||
|
הדרך בזה שתקח המורה הראשון לכל החלקים ותקח מהמורה החלקים ההם והעולה הוא השמור | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ערוך המורה על המספר המונח השני וחלק העולה על השמור והנה המספר המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה שיהיו ב' חמשיות וג' רביעיות ושלישית מספר מה עשרים ורצינו לדעת כמה כל המספר | ||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה המורה לכל אלו החלקים הוא ס' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
לקחנו ממנו אלו החלקים ועלה פ"ט והוא השמור | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ערכנו המורה על עשרים ועלה י"ב מאות | ||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקנום על השמור ועלה י"ג שלמים ומ"ג חלקים מפ"ט באחד שלם וככה המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תרצה תוכל לבחון זה | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והיה זה כן לפי שיחס ב' חמישיות ס' אל ס' כיחס ב' חמישיות המספר הנעלם אל המספר הנעלם | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן יתבאר שיחס ג' רביעיות ס' אל ס' כיחס ג' רביעית המספר הנעלם אל המספר הנעלם | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ושיחס שלישית ס' אל ס' כיחס שלישית המספר הנעלם אל המספר הנעלם | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר קבצנו הנה יהיה יחס כל החלקים האלו הלקוחים מס' אל ס' כמו יחס כל החלקים האלו הלקוחים מהמספר הנעלם אל המספר הנעלם | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
אם כן יחס פ"ט אל ס' הוא כיחס עשרים אל המספר הנעלם | ||||||||||||||||||||||||||||||||||||||||||||||||
|
אם כן שטח ס' שהוא השני בעשרים שהוא השלישי כמו שטח פ"ט שהוא ראשון במספר הנעלם | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והקש על זה | ||||||||||||||||||||||||||||||||||||||||||||||||
|
שאלה חלקים כמה מונחים ממספר נעלם מוסיפים על חלקים מונחים שניים מהמספר הנעלם מספר מונח כמה כל המספר | ||||||||||||||||||||||||||||||||||||||||||||||||
|
קח המורה אל כל החלקים וקח [719]ממנו כל החלקים המונחים הראשונים ושמור | ||||||||||||||||||||||||||||||||||||||||||||||||
|
גם קח[720] ממנו החלקים המונחים השניים והוא השמור השני | ||||||||||||||||||||||||||||||||||||||||||||||||
|
הוצא השמור השני מהשמור הראשון והנשאר הוא השמור המתוקן | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ערוך המורה על המספר המונח וחלק העולה על השמור המתוקן והעולה הוא המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה שיהיו ג' שביעיות וד' חמישיות המספר הנעלם מוסיפים על ב' שלישיות ורביעית המספר הנעלם עשרים ורצינו לדעת כמה המספר | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה המורה לכל אלו החלקים הוא ת"כ | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וג' שביעיותיו עם ד' חמישיותיו הוא תקי"ו | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וב' שלישיות ת"כ ורביעיתיו הם שפ"ה | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה החלקים הראשונים מוסיפים על החלקים השניים מספר קל"א | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ערכנו המורה על עשרים וחלקנו העולה על קל"א ועלה ס"ד שלמים וי"ו חלקים מקל"א באחד שלם וככה המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תרצה תוכל לבחון זה | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והיה זה כן לפי שיחס החלקים הראשונים מקובצים הלקוחים מת"כ אל ת"כ[721] כיחס החלקים הראשונים הלקוחים מהמספר הנעלם אל המספר הנעלם | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר המירונו הנה יחס החלקים הראשונים הלקוחים מת"כ אל החלקים הראשונים הלקוחים מהמספר הנעלם[722] כיחס ת"כ אל המספר הנעלם | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וכזה התבאר שיחס החלקים השניים הלקוחים מת"כ אל החלקים השניים הלקוחים מהמספר הנעלם הוא כיחס ת"כ אל המספר הנעלם | ||||||||||||||||||||||||||||||||||||||||||||||||
|
אם כן יחס החלקים הראשונים הלקוחים מת"כ אל החלקים הראשונים הלקוחים מהמספר הנעלם הוא כמו יחס החלקים השניים[723] הלקוחים מת"כ[724] אל החלקים השניים הלקוחים מהמספר הנעלם | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר המירונו הנה יחס החלקים הראשנים[725] הלקוחים מת"כ אל החלקים השניים הלקוחים מת"כ הוא כמו יחס החלקים הראשונים הלקוחים מהמספר הנעלם אל החלקים השניים הלקוחים מהמספר הנעלם | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר הבדלנו הנה יחס החלקים הראשונים הלקוחים[726] מת"כ אצל יתרונו על החלקים השניים הלקוחים מת"כ שהוא קל"א הוא כיחס החלקים הראשונים הלקוחים מהמספר הנעלם אצל העשרים שהוא יתרון על החלקים השניים הלקוחים מהמספר הנעלם | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר המירונו הנה יחס החלקים הראשונים הלקוחים מת"כ אל החלקים [727]הראשונים הלקוחים מהמספר הנעלם הוא כמו יחס קל"א אל עשרים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וכבר היה יחס החלקים הראשונים הלקוחים מת"כ אל החלקים הראשונים הלקוחים מהמספר הנעלם כיחס ת"כ אל המספר הנעלם | ||||||||||||||||||||||||||||||||||||||||||||||||
|
אם כן יחס ת"כ אל המספר הנעלם כיחס קל"א אל עשרים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
אם כן שטח ת"כ בעשרים הוא כמו שטח קל"א במספר הנעלם | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והקש על זה | ||||||||||||||||||||||||||||||||||||||||||||||||
Pricing Problems - Find the Price |
|||||||||||||||||||||||||||||||||||||||||||||||||
|
שאלה ערך מספר מונח ממסחר[728] מה כמה ערך המספר מונח שני מהמסחר ההוא | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ערוך הערך המונח על המספר המונח השני וחלק על המספר המונח ראשון וככה המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה שיהיה ערך י"א מדות תבואה ז' די' ותרצה לדעת כמה ערך ט"ו מדות תבואה | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ערוך ז' על ט"ו וחלק העולה על י"א ויעלה ט' שלמים וו' חלקים מי"א באחד וככה המבוקש רצוני שערך[729] ט"ו מדות הוא ט' די' וו' חלקים מי"א[730] בדינ' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והיה זה כן לפי שיחס המסחר הראשון אל המסחר השני כיחס הערך הידוע אל הערך הנעלם וזה מבואר בנפשו | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ולזה יהיה שטח מספר המסחר השני במספר הערך הידוע כמו שטח המספר המסחר הראשון בערך הנעלם | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והקש על זה | ||||||||||||||||||||||||||||||||||||||||||||||||
Conversion: day-hours, liṭra-dinar-pašuṭ |
|||||||||||||||||||||||||||||||||||||||||||||||||
| The method of converting given parts of the day into hours and fractions of hours, converting given parts of the liṭra, which is 20 dinar, into dinar and pešuṭim and fractions of pešuṭim, and similar things that have known parts: | דרך השבת חלקים מונחים מיום אל שעות ושברי שעה והשבת חלקים מונחים מן הליטרא שהוא כ' די' אל הדינרין והפשיטין ושברי הפשיטין ומה שידמה לזה מהדברים אשר הם בעלי חלקים ידועים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והמשל שרצינו לדעת כמה מן השעות ושברי השעה יהיו בפ"ג חלקים מק"ט[731] ביום | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה ידענו שמספר שעות היום הוא כ"ד | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה כיחס פ"ג אל ק"ט כן יחס מספר השעות הנעלם אל כ"ד | ||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה נערוך כ"ד על פ"ג ונחלק על ק"ט והנה המבוקש והוא י"ח שעות ונ"א ראשונים וי"ב שניים בקירוב | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם היה משלינו זה בליט' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה ידענו שמספר דינרי הליטרא הם עשרים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וכיחס פ"ג אל ק"ט כן יחס הנעלם [732]אל עשרים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה נכה עשרים בפ"ג ונחלק על ק"ט ויצא ט"ו די' וק"ו חלקים מק"ט בדינר | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וכבר תוכל לדעת כמה הם מן הפשוטים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
בשתערוך י"ב על ק"ו ותחלק על ק"ט ויצא י"א פשוטים וע"ג חלקים מק"ט בפשוט שהם ב' שלישיות פשוט אחד בקירוב | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ובזה תנהיג מה שידמה לזה | ||||||||||||||||||||||||||||||||||||||||||||||||
| The method of converting fractions of different types into one type [of fraction]: | דרך השבת חלקים ממינים מתחלפים חלקים ממין אחד | ||||||||||||||||||||||||||||||||||||||||||||||||
|
קח המורה הראשון שימנוהו כל המורים על השברים ההם המתחלפים וממנו תקח החלקים ההם והעולה הם חלקים מהמורה | ||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה אם רצית להשיב ג' שביעיות וד' חלקים מי"ז שברים ממין אחד | ||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה תקח מהמורה שהוא קי"ט כל אלו החלקים והיה העולה הוא ע"ט חלקים מקי"ט[733] באחד | ||||||||||||||||||||||||||||||||||||||||||||||||
| The method of converting fractions of one type into a unit fraction: | דרך השבת החלקים ממין אחד חלק אחד | ||||||||||||||||||||||||||||||||||||||||||||||||
|
חלק המורה על החלקים ההם על מספר החלקים ההם והעולה הוא המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה אם רצית להשיב ג' שביעיות חלק אחד | ||||||||||||||||||||||||||||||||||||||||||||||||
|
תחלק שבעה על ג' ויהיה העולה ב' ושליש והוא חלק אחד מב' ושליש בדבר ההוא אשר רצית לקחת ג' שביעיותיו | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והקש על זה | ||||||||||||||||||||||||||||||||||||||||||||||||
Motion Problems |
|||||||||||||||||||||||||||||||||||||||||||||||||
|
שאלה המתנועע תנועה שוה הולך[734] בזמן מונח שעור מונח[735] מן הדרך כמה ילך מן הדרך בזמן מונח שני | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ערוך מספר הזמן המונח השני על השעור המונח מן הדרך והעולה חלק על מספר הזמן המונח ויצא לך המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם היו שעות באחד מן הזמנים או בשניהם השב מספר הזמן לשעות והשעות תהיינה לאחדים שלמים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והמשל שיתנועע המתנועע בי"ג ימים ז' שיעורים מן הדרך ול"ו ראשונים נ"ז שניים ורצינו לדעת כמה מן הדרך ילך בג' ימים וי"ז שעות ונ"ב ראשונים י"ו שניים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה נשיב הימים שעות | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ויהיה הזמן[736] הראשון המונח שי"ב | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והזמן השני פ"ט שלמים ונ"ב ראשונים י"ו שניים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ערכנו ז' [737]שלמים ל"ו ראשונים נ"ז שניים על פ"ט שלמים ונ"ב ראשונים י"ו שניים וחלקנו העולה על שי"ב ועלה ב' שלמים י' ראשונים נ"ו שניים והוא מספר השעורין שהלך מן הדרך בזמן המונח השני | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והיה זה כן לפי שהתנועה השוה תהיה בזמנים שוים שעורים שוים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ולזה הוא מבואר שיחס הזמן אל הזמן הוא יחס התנועה אל התנועה | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וכבר תפול השאלה בהפך זה ר"ל שישאל השואל במתנועע תנועה שוה ההולך שעור מונח מן הדרך בזמן מונח בכמה מן הזמן ילך שעור מונח שני מן הדרך | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ואז תערוך השעור המונח השני מן הדרך על הזמן המונח וזה[738] בשתשיב הימים שעות להקל מעליך והעולה תחלק על השעור המונח הראשון מן הדרך והנה המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והמשל שילך המתנועע ז' שעורים מן הדרך ול"ו ראשונים נ"ז שניים בי"ג[739] ימים ורצינו לדעת בכמה מן הזמן ילך ג' שעורים מן הדרך | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ערכנו ג' שעורים על שי"ב שלמים וחלקנו העולה על ז' שלמים ל"ו ראשונים נ"ז שניים ועלה קכ"ב שעות ונ"ד ראשונים וז' שניים והוא הזמן הנעלם | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה מבואר מהסבה הקודמת בעינה | ||||||||||||||||||||||||||||||||||||||||||||||||
Motion Problems - Pursuit |
|||||||||||||||||||||||||||||||||||||||||||||||||
|
שאלה אם היו שני מתנועעים תנועה שוה והיה האחד יותר מהיר במהלכו מן האחר ומרחק המהיר מהמתוני לאחריו מדה[740] מונחת מן הדרך בכמה מן הזמן ישיג המהיר המתוני | ||||||||||||||||||||||||||||||||||||||||||||||||
|
חלק המדה המונחת על יתרון מהלך מהיר על המתוני לשעה והעולה בידך הם השעות וחלקי השעה אשר ישיג בהם המהיר המתוני | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והמשל שיהיה מהלך מהיר לשעה אחת ב' שעורים ול"ז שניים ויהיה מהלך המתוני לשעה אחת ל' ראשונים כ"ד שניים והיה מרחק המתוני מהמהיר לפניו כ"ט שעורים ומ"ה ראשונים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה יתרון מהלך המהיר על מהלך המתוני לשעה הוא שעור אחד ול' ראשונים וי"ג שניים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
[741]חלקנו עליו המרחק המונח ועלה י"ט שעות מ"ז ראשונים ט' שניים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה מבואר הסבה עם מה שקדם מהדברים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
|
|||||||||||||||||||||||||||||||||||||||||||||||||
|
שאלה הכלי המלא המונח יש לו נקבים מתחלפים יצא מהאחד מהם[742] כל מה שבכלי בזמן מונח | ||||||||||||||||||||||||||||||||||||||||||||||||
|
חקור תחלה על מה שיצא מכל אחד מהנקבים ההם בשעה אחת וקבץ הכל | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וראה יחסו אל מלא הכלי וכפי יחסו אליו כן יחס השעה אל הזמן שיצא בו מלא הכלי | ||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה שהחבית יש בו נקבים מתחלפים בנקב האחד יצא מלא החבית בג' ימים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה בנקב הראשון יצא מהחבית בשעה אחת חלק אחד מע"ב חלקים במלא החבית | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ובנקב השני חלק מק"כ[744] | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ובנקב השלישי חלק מכ' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ובנקב הרביעי חלק מי"ב | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר קבצנו זה כלו יהיה מה שיצא מכלם יחד בשעה אחת[745] נ"ו חלקים מש"ס חלק במלא החבית | ||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקנו ש"ס על נ"ו ועלה ו' שלמים כ"ה ראשונים מ"ג שניים[746] | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה הזמן שיורק בו הכלי הוא ו' שעות כ"ה ראשונים מ"ג שניים בקירוב | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וסבת זה מבוארת | ||||||||||||||||||||||||||||||||||||||||||||||||
Pricing Problems |
|||||||||||||||||||||||||||||||||||||||||||||||||
|
שאלה ערך המסחר[747] המונח מספר מונח מן הדינרין כמה ערך חלקים מה מונחים ממנו | ||||||||||||||||||||||||||||||||||||||||||||||||
|
קח המורה אל כל החלקים וקח ממנו החלקים ההם והעולה הוצא ערכו וכפול אותו על מספר המסחר וחלק העולה על המורה וככה המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה שיהיה ערך דינר זהב כ"ה דינרי' ורצינו לדעת כמה ערך הדי' עם חציו וב' שביעיותיו וג' רביעיותיו | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה המורה לכל אלו השברים הוא כ"ח | ||||||||||||||||||||||||||||||||||||||||||||||||
|
לקחת ממנו אלו החלקים ועלה ע"א[748] | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ערכת ע"א על כ"ה ועלה אלף תשע"ה שהוא ערך החלקים הלקוחים מהמורה | ||||||||||||||||||||||||||||||||||||||||||||||||
|
[749]ערכת זה המספר על מספר המסחר שהוא אחד וחלקת העולה על כ"ח ועלה ס"ג שלמים וי"א חלקים מכ"ח באחד וככה הערך ר"ל ס"ג די' [750]וי"א חלקים מכ"ח בדינר | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תרצה תוכל לבחון זה | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והיה זה כן לפי שיחס החלקים הלקוחים מהמורה אל החלקים הלקוחים[751] מדינרי זהב הוא כיחס המורה אל אחד שהוא מספר המסחר[752] | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
ולזה יהיה יחס ערך החלקים הלקוחים מהמורה אל ערך החלקים הלקוחים מדינרי זהב כיחס המורה אל אחד | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
לפי שהוא מבואר שיחס המסחר אל המסחר הוא יחס הערך[753] אל הערך | ||||||||||||||||||||||||||||||||||||||||||||||||
|
אם כן שטח מספר ערך החלקים הלקוחים מהמורה באחד שוה לשטח מספר ערך החלקים הלקוחים מן הדינר במורה | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
והקש על זה | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם היה המסחר מספר מה בזה בעינו יתבאר | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והמשל שיהיה ערך ז' מדות תבואה כ"ה די' ורצינו לדעת כמה ערכם עם חציים וב' שביעיותיהם וג' רביעיותיהם | ||||||||||||||||||||||||||||||||||||||||||||||||
|
לקחנו אלו החלקים מהמורה שהוא כ"ח ועלה ע"א | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה ערכם רנ"ג די' וד' שביעיות הדינר | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ערכת אותם על מספר המסחר שהוא ז' וחלקת העולה על המורה שהוא כ"ח ועלה ס"ג די' וי"א חלקים מכ"ח בדינר | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
והקש על זה | ||||||||||||||||||||||||||||||||||||||||||||||||
Purchase Problems - Equal Amounts |
|||||||||||||||||||||||||||||||||||||||||||||||||
|
שאלה הסוחר מוכר מסחרים מתחלפי[754] הערכים כמה שיהיו ורצה הקונה לקנות בסך מה מן הממון מדה שוה מכל אחד מהמסחרים[755] | ||||||||||||||||||||||||||||||||||||||||||||||||
|
הדרך בזה שתקבץ ערכי המדה האחת לכל המסחרים והעולה שמור | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וכמו יחס הסך שבידו אל השמור קח מהמדה מכל אחד מהמסחרים[756] והנה המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה שימכור הסוחר מד' סמים ערך הסם הראשון ז' פשיטין הליטרא | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה [757]בג' די' וד'[758] פשי' יקח ליטרא מכל אחד | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה יחס ג' די' אל ג' די' וד' פשי' הוא ט' עשיריות וככה יקח מכל סם וסם ר"ל ט' עשיריות ליטרא | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ויהיה ערך הכל ג' די' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תרצה תוכל לבחון זה | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והיה זה כן לפי שיחס מה שיקח מכל ליטרא מהם אל הליטרא כיחס ערך מה שיקח מכל ליטרא מהם אל ערך הליט' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ואולם יחס מה שיקח מליט' באחד מהם אל הליט' הוא כיחס מה שיקח מליט' מכל אחד מהם אל הליטרין | ||||||||||||||||||||||||||||||||||||||||||||||||
|
יהיה אם כן יחס ערך מה שיקח מאחד מהם אל ערך הליטר' כיחס ערך מה שיקח מכל אחד מהם אל ערך הליט' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר קבצנו הנה יחס מה שיקח מכלם יחד אל מספר ליטראות כמספרם כיחס ערך מה שיקח מכלם יחד אל ערך מספר ליטראות כמספרם | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והקש על זה | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ומזאת השאלה יתבאר אם רצה השולחני לקנות בדינר זהב מטבעות מתחלפות הערכים ורצה לקחת מכל מטבע מהם מספר שוה כמה יקח מכל אחד ואחד | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והמשל שיהיה ערך המטבע האחד ג' די' הזהוב וערך השני ה' די' הזהוב | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה בשליש הזהוב יקנה די' ממטבע האחד | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ובחמישית הזהוב יקנה די' מהמטבע האחר[760] | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ובשביעית הזהוב יקנה די' מהמטבע האחר | ||||||||||||||||||||||||||||||||||||||||||||||||
|
אם כן בשלישית הזהוב וחמישיתו ושביעיתו יקח די' מכל אחד מהם | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וכמו יחס הזהוב אל אלו החלקים כן יחס מה שיקח מכל אחד מהם אל הדינר | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וכזה יקח מכל אחד מהם דינר ול"ד חלקים מע"א בדי' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וכפי זה תקיש במה שידמה לזה | ||||||||||||||||||||||||||||||||||||||||||||||||
|
שאלה הסוחר מוכר מסחרים מתחלפי הערכים כמה המספר המעט מן המדות שילקח מאחד אחד מהם ויהיה ערך מה שילקח מזה כערך מה שילקח מזה | ||||||||||||||||||||||||||||||||||||||||||||||||
|
הדרך בזה שתכתוב ערכי המסחרים[761] זה אחר זה בטור אחד | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וכנגדם תמיד בטור אחד המספר האחד תחת האחר [762]וכן תעשה לכל המספרים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ואחר זה תקח קטני המספרים על יחס אלו והעולה בידך תחת כל אחד מן הערכין הוא מספר המדות שילקח מהמסחר[763] ההוא | ||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה שימכור הסוחר מארבעה סמים ערך הסם האחד ב' די' הליט' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה תכתוב בטור אחד ערכי הליטראות והוא ב' ג' י"ב כ' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ובטור אחר תחתיו תמיד הענין על זה הסדור[764] שאזכיר רצוני שתכתוב ב' ג' ותחת ג' ב' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וגם כן הנה תכתוב על זה הדרך תחת ג' י"ב ותחת י"ב ג' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וגם כן הנה תכתוב תחת י"ב כ' ותחת כ' י"ב | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||
|
ואחר כן תקח קטני המספרים המתיחסים בכמו זה היחס ר"ל מיחס ג' אל ב' ומיחס י"ב אל ג' ומיחס כ' אל י"ב והם לפי מה שהתבאר מאקלידס מספרי ל' כ' ה' ג' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה שיחס ל' אל כ' כיחס ג' אל ב' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ויחס כ' אל ה' כיחס י"ב אל ג' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ויחס ה' אל ג' כיחס כ' אל י"ב | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ולזה תקח מן הסם אשר ערכו ב' די' ל' ליטרין והוא המספר הנכחי לו | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ומהסם אשר ערכו ג' די' הליט' תקח כ' ליטראות | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ומהסם אשר ערכו י"ב די'[765] תקח ה' ליטראות | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ומן הסם אשר ערכו כ' די' תקח ג' ליטראו' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ויהיה ערך מה שתקח מכל אחת מהם שוה | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והקש על זה | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וראוי שתשמור בזה הדרך הנזכר אם היה ערך אחד הסמים מספר הפשוטים שתשיב ערך הסמים הנשארים לפשוטים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ומזה תקיש[766] במה שידמה לזה | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והיה זה כן לפי שיחס ל' אל כ' הוא כיחס ג' אל ב' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
אם כן שטח ל' בב' הוא כמו שטח כ' בג' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
אבל שטח ל' בב' שוה לערך ל' ליטראו' מב' די' הליטר' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ושטח כ' בג' שוה לערך כ' ליטראות מג' די' הליטרא | ||||||||||||||||||||||||||||||||||||||||||||||||
|
אם כן ערך ל' ליטראות מב' די' הליט' שוה לערך כ' ליט' מג' די' הליטרא | ||||||||||||||||||||||||||||||||||||||||||||||||
|
[767]וכזה התבאר שערך כ' ליטר' מג' די' הליט' שוה לערך ה' ליט' מי"ב די' הליט' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ושערך ה' ליטראו' מי"ב די' הליט' הוא כמו ערך ג' ליטראו' מכ' די' הליט' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והקש על זה | ||||||||||||||||||||||||||||||||||||||||||||||||
|
שאלה הסוחר מוכר מסחרים מתחלפי הערכים ובא הקונה לקנות מדה אחת מכלם ויהיה ערך מה שיקח מזה שוה לערך מה שיקח מזה כמה מן המדה יקח מכלם וכמה ערך המדה ההיא | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ראוי שתקח קטני המספרים שילקח מכל אחת מהן מהמדות ויהיה הערך שוה וכאשר ישלם לך זה קבץ מספריהם והוא השמור והשמור הם חלקי המדה | ||||||||||||||||||||||||||||||||||||||||||||||||
|
לקח מכל אחד מהם מאלו החלקים כמו המספר אשר תחתיו והנה המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
ואולם ערך המדה ההיא תדעהו בשתקבץ ערכי קטני המספרים ההם[768] כל אחד מגילו והוא ערך המקובץ חלקהו על השמור והנה המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה שימכר הסוחר משלשה סמים ערך האחד ז' פשי' הליטר' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה קטני המספרים שילקחו מאחד אחד מהם ויהיה הערך שוה הם מספרי כ' י"ד ז' והנה מקובצם הוא מ"א והם חלקי הליט' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וילקח מאלו החלקים מהסם אשר ערכו ז' פשי' הליט' כ' חלקים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ומהסם אשר ערך הליט' ממנו י' פשי' ילקחו י"ד חלקים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ומהסם אשר ערך הליט' ממנו כ' פשי' ילקחו ז' חלקים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה הכל[769] מ"א חלקים שהיא הליט' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ואולם ערך הליט' הנה תקח ערך מספרי כ' י"ד ז' כל אחד מהנכחי לו ותקבץ הכל והנה הכל הוא ל"ה די' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקת ל"ה די' על מ"א ועלה י' פשו' וי' חלקים[770] ממ"א בפשוט והוא המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והקש על זה | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והיה זה כן כי מפני שערך כ' ליט' מהערך הקטן הוא כערך י"ד ליט' מהערך האמצעי וכמו ערך ז' ליט' מערך הגדול | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה יחס כ' חלקים [771]ממ"א בליט' אל כ' ליט' הוא כמו יחס י"ד חלקים ממ"א בליט' אל י"ד ליטרי' וכמו יחס ז' חלקים ממ"א בליט' אל ז' ליט' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ולזה יהיה יחס ערך כ' חלקים ממ"א בליט' אל ערך כ' ליט' כמו יחס ערך י"ד חלקים ממ"א בליט' אל ערך י"ד ליט' וכמו יחס ערך ז' חלקים ממ"א בליט' אל ערך ז' ליט' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר המירונו הנה יחס ערך כ' חלקים ממ"א בליט' מהערך הראשון אל י"ד חלקים ממ"א בליט' מהערך השני הוא כמו יחס ערך כ' ליטר' מהערך הראשון אל ערך י"ד ליטר' מהערך השני | ||||||||||||||||||||||||||||||||||||||||||||||||
|
אבל ערך כ' ליטראות מהערך הראשון הוא כמו ערך י"ד ליטר' מהערך השני | ||||||||||||||||||||||||||||||||||||||||||||||||
|
אם כן ערך כ' חלקים ממ"א בליטרא מהערך הראשון הוא כמו ערך י"ד חלקים ממ"א בליטרא מהערך השני[772] | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וכזה התבאר שערך י"ד חלקים ממ"א בליט' מהערך השני הוא כמו ערך ז' חלקים ממ"א בליט' מהערך השלישי | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והקש על זה | ||||||||||||||||||||||||||||||||||||||||||||||||
Purchase Problems - Unequal Amounts |
|||||||||||||||||||||||||||||||||||||||||||||||||
|
שאלה הסוחר מוכר שני מסחרי' מתחלפי הערכים ורצה הקונה לקנות מדה אחת משניהם יהיה ערכה מספר מה מוסיף על הערך הקטן ומחסיר מהערך הגדול | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
ראוי שתקח מספר יתרון המוסיף על המחסיר והם חלקי המדה | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ואחר תקח מספר חסרון המחסיר[773] והוא יהיה מספר החלקים שיקח מהמוסיף ומספר יתרון המוסיף הוא יהיה מספר החלקים שיקח מהמחסיר | ||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה שיהיה הסוחר מוכר משני סמים ערך הסם האחד י"ז פשי' הליט' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה יתרון המוסיף[774] על המחסיר הוא ז' והם חלקי המדה | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה חסרון המחסיר מי"ט הוא שנים והם החלקים מחלקי המדה שיקח מהסם אשר ערכו מוסיף | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה יתרון המוסיף הוא חמשה והם חלקים שיקח מהסם אשר ערכו מחסיר | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ויהיה ערך המדה י"ט פשי' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והקש על זה | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והיה זה כן לפי שבכאן שלשה מספרים מתחלפים והם מספרי י"ז י"ט כ"ד | ||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה יהיה שטח כ"ד בשנים שהוא יתרון האמצעי על הקטן עם שטח י"ז בחמשה שהוא יתרון הגדול על האמצעי ימנהו י"ט שהוא האמצעי במספר אחדי יתרון הגדול על הקטן שהוא ז' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
אם [775]כן ב' ליט' מערך כ"ד פשי' עם ה' ליט' מערך י"ז פשי' יהיה מקובץ הערכים שוה לשטח ז' בי"ט | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ומספר הליט' ז' אם כן כל אחת תעמוד בי"ט פשי' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וגם כן הנה יחס שתי שביעיות ליט' אל ב' ליט' כיחס חמשה שביעיות ליט' אל חמשה ליט' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ולזה יהיה יחס ערך שתי שביעיות ליטרא מהערך הגדול אל ערך שתי ליטראות מהערך הגדול כיחס ערך חמשה שביעיות ליטרא מהערך הקטן אל ערך חמשה ליטראות מהערך הקטן[776] | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ולזה יהיה יחס ערך שתי שביעיות ליט' מהערך הגדול וחמשה שביעיות ליטר' מהערך הקטן אל ערך ב' ליט' מהערך הגדול וחמשה ליטראות מהערך הקטן כיחס ערך שתי שביעיות ליט' מהערך הגדול אל ערך שתי ליט' מהערך הגדול | ||||||||||||||||||||||||||||||||||||||||||||||||
|
אבל יחס ערך שתי שביעיות ליט' מהערך הגדול אל שתי ליט' מהערך הגדול והוא שביעית הערך | ||||||||||||||||||||||||||||||||||||||||||||||||
|
אם כן יחס ערך שתי שביעיות ליט' מהערך הגדול וה' שביעיות ליט' מהערך הקטן אל ערך ב' ליט' מהערך הגדול וה' ליט' מהערך הקטן הוא שביעית הערך | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וכבר התבאר שזה הערך כלו הוא שטח ז' בי"ט | ||||||||||||||||||||||||||||||||||||||||||||||||
|
אם כן ערך זאת הליט' הוא שביעית שטח ז' בי"ט שהוא י"ט פשי' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והקש על זה | ||||||||||||||||||||||||||||||||||||||||||||||||
|
שאלה הסוחר מוכר מסחרים מתחלפי הערכים כמה שיהיו ובא הקונה לקנות מדה אחת מכלם יהיה ערכה מוסיף על הערך הקטן ומחסיר מן הערך הגדול כמה מן המדה יקח מאחד אחד מהם | ||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה ראוי שתזוג עם כל אחד מהמחסירים[777] אחד מן המוסיפים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם לא יספיקו המוסיפים או המחסירים לפי שהיה מספר המוסיפים יתר או פחות הנה תזוג עם כל אחד מהנשארים ממקביליהם המספר אשר ערכו יותר קרוב אל הערך המבוקש כדי שימעט המספר המתר | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ואחר תנהיג מכל זוג וזוג על הצד הקודם והנקבץ מיתרון כל אחד מהמוסיפים על זוגו הם חלקי הליט' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם היה שם מסחר יהיה ערכו כמו[778] הערך המבוקש הנה זה המסחר אין זוג לו ולזה תקח ממנו חלק אחד או חלקים כפי מה שתרצה ותוסיף על מספר החלקים הנקבצים והנקבץ יהיו חלקי [779]הליט' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה שיהיה הסוחר מוכר מז' סמים[780] ערך הסם הראשון ג' פשי' הליט' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה המוסיפים הם שנים הסם אשר ערכו י"ט פשי' הליט' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והמחסירים הם ארבעה והם הסם אשר ערכו ג' פשי' ואשר ערכו ה' פשי' ואשר ערכו ח' פשי' ואשר ערכו י"א פשי' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה נשים ג' פשי' גיליי לי"ט פשי' וה' פשי' גיליי לכ"ח פשי' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ומפני שנשארו עוד סמים במחסירים ולא נשאר מהמוסיפים נקח מהמוסיפים[783] היותר קרוב אל ט"ו שהוא הערך המבוקש והוא י"ט ונזווג[784] י"ט עם כל אחד מהנשארים מהמחסירים כמו שתראה בזאת הצורה | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||
|
וכבר ידענו איך נקח ליט' מכל זוג וזוג מאלו יהיה ערכה ט"ו פשי' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ולזה נקח מסם ג' ד' חלקים מי"ו בליט' ומסם י"ט י"ב חלקים מי"ו בליט' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ולקח גם כן מסם ה' י"ג חלקים מכ"ג בליט' ומסם כ"ח י'[785] חלקים מכ"ג בליט' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ונקח גם כן מסם ח' ד' חלקים מי"א בליטרא ומסם י"ט ז' חלקים מי"א בליטרא[786] | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ונקח גם כן מסם י"א ד' חלקים מח' בליט' ומסם י"ט ד' חלקים מח' בליט' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה מספר כל אלו החלקים הוא נ"ח | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ונוסיף שני חלקים בעבור ט"ו שאין לו גיליי והנה ס' והם חלקי הליט' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
נקח מהם מסם[787] ג' פשי' ד' חלקים ומסם ה' י"ג חלקים[788] ומסם ח' ד' חלקים ומסם י"א ד' חלקים ומסם ט"ו שני חלקים ומסם י"ט כ"ג חלקים ומסם כ"ח י' חלקים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ויהיה ערך הליט' ט"ו פשי' והוא המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והיה זה כן לפי שהוא מבואר ממה שקדם שד' ליט' מערך ג' עם י"ב ליטראות מערך י"ט ערכם כמו שטח י"ו בט"ו | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ובזה התבאר הענין בכל שני מספרים גיליים מאלו | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והוא מבואר שב' ליט' מערך ט"ו ערכם גם כן כמו שטח ב' בט"ו | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר קבצנו הכל הנה ערך כל אלו הליט' במספרם [789]מוכה בט"ו אשר הוא כמו שטח ס' בט"ו | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ואולם יחס חלק מס' בליט' אל הליט' כמו יחס ערך חלק מס' בליטרא[790] אל ערך הליט' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ויתבאר על צד הבאור הקודם שיחס ערך כל החלקים מקובצים אל ערך מקובץ הליט' שהוא כמו שטח ס' בט"ו הוא כיחס אחד אל ס' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ולזה יהיה ערך החלקים בכללם ט"ו פשי' שהוא אחד מס' בשטח ס' בט"ו | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והקש על זה | ||||||||||||||||||||||||||||||||||||||||||||||||
Payment Problem |
|||||||||||||||||||||||||||||||||||||||||||||||||
|
שאלה איש אחד שכר איש אחר מספר מונח מהממון שיעשה מלאכתו מספר מונח מן הימים וזאת המלאכה אשר יעשה ישכור לו בכל יום מאלו הימים מספר מונח מן האנשים ינהיג כל אחד מספר מונח מהבהמות תשא כל אחד מהם מספר מונח מן המדות ותלך כל אחת מהן מספר מונח מן הדרך ושנה השכיר בקצת המספרים או בכלם כמה שכירותו | ||||||||||||||||||||||||||||||||||||||||||||||||
|
קח המספר המורכב מכל המספרים שהתנה ושמור | ||||||||||||||||||||||||||||||||||||||||||||||||
|
גם קח המספר המורכב מכל המספרים שהשלים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וכיחס השמור אל זה המספר המורכב כן יחס השכירות שהתנה לתת לו אל מה שהוא חייב לתת לו | ||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה ששכר ראובן את שמעון י' ליט' שיעשה מלאכתו ט' ימים וזאת המלאכה ישכור לו בכל יום י"ג מן האנשים ינהיג כל אחד מהם ז' מהבהמות תשא כל אחת מהם ט"ו מהמדות ותלך ו' פרסאות והוא שכר לו ח' ימים י"ז מן האנשים ותנהיג כל אחד מהם ששה מהבהמות ונשאה כל אחד מהם י"א מן המדות והלכה ז' פרסאות | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה המספר המורכב ממספרים שהתנה שהם ט' י"ג ז' ט"ו ו' והוא ע"ג אלפים ותש"י והוא השמור | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והמספר המורכב מהמספרים שהשלים שהם ח' י"ז ו' י"א ז' הוא ס"ב אלפים ותתל"ב | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה יחס י' ליט' אל מה שהוא חייב לו כיחס השמור אל ס"ב אלפים ותתל"ב | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תערוך י'[791] ליט' שהוא הראשון על הרביעי שהוא ס"ב אלפים תתל"ב ותחלק העולה על השמור יצאו לך הליטראות וחלקי הליט' שהוא חייב לו הם ח' ליט' וחצי ואלף וז' מאות ופ"ה חלקים מס"ב אלפים ותתל"ב בליט' שהוא ה'[792] [793]פשי' ונ"ט אלפים תת"נ חלקים מע"ג אלפים תש"י בפשוט | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
והיה זה כן לפי שיחס מה שהוא חייב לו אל מה שהתנה הוא כיחס מה שעשה אל מה שהתנה לעשות | ||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
ויחס מה שעשה אל מה שהתנה לעשות הוא מחובר מיחסי המספרים שהתנה אל גיליהם שהשלים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה שיחס המחובר כבר התבאר שהוא כמו יחס המספר המורכב מהמספרים שהתנה אל המספר המורכב מהמספרים שהשלים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והקש על זה | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ומזה הבאור התבאר אם התנה אדם לאדם למלאת ממסחר מה כלי ארכו מספר מונח ורחבו מספר מונח ועמקו מספר מונח במספר מה מן הממון ומלא לו כלי אחר מתחלף הרחקים לכלי אשר התנה כמה חייב לו | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה שכמו יחס המספר המורכב מהשלשה רחקים אשר התנה אל המספר המורכב מהשלשה רחקים אשר השלים כן יחס הערך שהתנה לו אל מה שהוא חייב לו | ||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן תשפוט במה שידמה לזה | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והמשל שמכר המוכר לקונה מלא כלי משמן בערך כ' די' ארך הכלי עשרה מדות ורחבו ט' ועמקו י"ב | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה המספר המורכב מהרחקים הקודמים הוא אלף ופ' והוא השמור | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והמספר המורכב מהמרחקים שהשלים הוא אלף וקכ"ב | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה כיחס השמור אל אלף וקכ"ב כן יחס כ' די' אל[794] מה שהוא חייב לו | ||||||||||||||||||||||||||||||||||||||||||||||||
Buy and Sell Problems |
|||||||||||||||||||||||||||||||||||||||||||||||||
|
שאלה ראובן קנה חלקים ככה מהמדה במספר ככה מהדינר ומכר חלקים ככה מהמדה במספר ככה מהדינר והיה הממון ככה ורצינו לדעת אם הרויח או הפסיד וכמה | ||||||||||||||||||||||||||||||||||||||||||||||||
|
הדרך בזה שתוציא סך דינרי ד' ערך המדה לכל אחד מהערכין | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ואחר כן יתבאר לך אם הפסיד או הרויח במדה וכמה מן הדינר | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ובזה יתבאר לך כמה סך מדות המסחר | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ערוך סך מדות המסחר על מה שהרויח במדה או הפסיד והעולה הוא סך הדינר שהפסיד או הרויח | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם היתה השאלה בהפך ר"ל שהרויח או הפסיד מספר ככה מהדינר ובאת לדעת כמה היה הממון [795]או המסחר | ||||||||||||||||||||||||||||||||||||||||||||||||
|
תחלק המספר מהדינר שהרויח בכל המסחר או הפסיד על מה שהרויח[796] במדה או הפסיד והעולה הוא סך מדות המסחר | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וערכם לפי מה שקנה הוא הממון | ||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון ראובן קנה ב' חמישיות המדה וג' שביעיותיה ז' די' וח' חלקים מי"א בדי' ומכר ד' תשיעותיה ח' די' וג' שביעיות הדינר | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה ערך המדה לפי מה שקנה ט' די' וק"ד חלקים משי"ט בדינר | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וערך המדה לפי מה שמכר י"ח דינרי' וכ"ז חלקים מכ"ח בדי' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ולזה היה סך מדות המסחר י' מדות וכ"א מאות ונ' חלקים מכ"ט מאות וע"ה במדה שהם פ"ו חלקים מקי"ט במדה | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה הרויח במדה ט' די' וה' אלפים ותש"א חלקים מח' אלפים תתקל"ב בדינר | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ערכנוהו על מדות המסחר והנה הרויח ק"ג די' וש"ע אלפים ומ' חלקים מאלף וס"ב אלפים ותתק"ח בדינר והם ג' אלפים וק"צ חלקים מט' אלפים קס"ג בדינר | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם אמר השו[אל][797] שהרויח ראובן או הפסיד בזה המסחר ק' די' ורצית לדעת כמה הממון | ||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה תחלק במשלינו זה[798] ק' די' על מה שהרויח במדה אחת שהוא ט' די' וה' אלפים תש"א חלקים מח' אלפים תתקל"ב בדינר והעולה תהיינה מדות המסחר[799] והם י'[800] מדות ול"ב אלפים וש"י חלקים מפ"ו אלפים ופ"ט חלקים במדה | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תערוך מדות המסחר על סך די' ערך המדה לפי מה שקנה יהיה העולה בידך הממון | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה כלו מבואר הסבה עם מה שקדם מהדברים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
[801]שאלה איש אחד קנה חלק מה או חלקים מה מהמדה או ליט' במספר מונח מהדינר ומכר חלקים אחרים מחסירים מן הראשון במספר ההוא בעינו מן הדינר והרויח ככה כמה הממון | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והמשל שקנה ראובן ב' חמישיות המדה וג' שביעיותיו ב' די' ומכר ד' תשיעיותיה בדינר | ||||||||||||||||||||||||||||||||||||||||||||||||
|
הדרך בזה שתקח המורה לכל אלו החלקים הן [802]מהמדה הן מהדינרין ואם קרה שיהיו בהם חלקים והנה המורה לכל אלו החלקים במשלנו הוא שט"ו והיא המדה | ||||||||||||||||||||||||||||||||||||||||||||||||
|
קח ממנו ב' חמישיותיו וג' שביעיותיו ויעלה רי"ו | ||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה אם כן בדי' יקנה מאלו החלקים ק"ח | ||||||||||||||||||||||||||||||||||||||||||||||||
|
גם קח ממנו ד' תשעיותיו ועלה ק"מ | ||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה אם כן בדי' יתן ע' מאלו החלקים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ולזה ירויח בדי' ל"ח חלקים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וכבר היה הריוח ק' די' שהוא ז' אלפים מאלו החלקים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה ראוי שנקח מספר יהיה יחסו אל ק"ח כיחס ז' אלפים אל ל"ח | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וכבר התבאר דרך לקיחתו | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם חלקת העולה על ע' תמצא מספר הממון עם מה שהרויח יחד | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם חלקת העולה על ק"ח שהוא סך חלקי הדינר לפי מה שקנה תמצא מספר הממון | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה מבואר הסבה עם מה שקדם מהדברים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם היתה השאלה בהפך ר"ל שהפסיד ק' די' בשקנה ד' תשיעיות המדה ב' די' ומכר ב' חמישיותיה עם ג' שביעיותיה ב' די' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
הדרך אחת אלה שכאשר תחלוק העולה על ע' יהיה לך מספר הממון | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תחלקהו על ק"ח יהיה לך הנשאר לו אחר ההפסד | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם שאל כמה המקח חלק העולה על שפ"ה שהם חלקי המדה והנה מדות המקח | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והקש על זה | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן אם לא היה מספר הדי' שוה | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ר"ל שקנה חלקים מה מהמדה בג' די' ומכר חלקים מה ממנה כ"ה די' והרויח או הפסיד ק' די' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
תוציא בדרך הקודם החלקים מהמורה שיקנה די' גם החלקים שימכר די' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ותמשיך המעשה על האופן הקודם ויצא לך הדרוש | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם היו החלקים אחדים ומספר הדי' מתחלף | ||||||||||||||||||||||||||||||||||||||||||||||||
|
משל זה שיקנה ד' חלקים מז' בליט' עם ה' חלקים מי"א בה ז' די' וח' חלקים מי"א בדי' וימכר אלו החלקים ט' די' וג' חלקים מי"ג די' והרויח ק' די' ושאל שואל כמה הממון או כמה המקח | ||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה הדרך שתקח המורה אל כל החלקים הן מהליט' והוא יהיה חלקי הליט' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ותדע סך החלקים שיקנה בדי' וסך החלקים שיתן בדי' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ותמשיך הענין על האופן הקודם הן לדעת מספר הממון הן לדעת מספר המקח | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם ישאל אדם כמה שעור בעל שעור מה שב' שביעיותיו עם ג' חמישיותיו מוסיפים על ד' תשיעיותיו שעור מה או מחסירים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה הדרך בזה מבוארת בתמונת מ"ט | ||||||||||||||||||||||||||||||||||||||||||||||||
|
[803]שאלה ראובן קנה חלקים ככה מהמדה במספר ככה מהדי' והיה הממון מספר ככה מן הדי' ומכר קצת המסחר חלקים ככה מהמדה במספר ככה מהדי'[804] וקצתו האחר מכר חלקים ככה מהמדה במספר ככה מהדי' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה מן המחוייב אם היתה השאלה צודקת שירויח בערך האחד ויפסיד בערך השני | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה תוציא ערך המדה לכל אחד מהערכים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ותראה כמה הפסיד במדה לערך האחד וכמה הרויח במדה לערך השני | ||||||||||||||||||||||||||||||||||||||||||||||||
|
[805]והנה כיחס ההפסד אל הריוח כן יחס מה שמכר לערך המותיר אל מה שמכר לערך המחסיר | ||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה יתבאר במעט עיון מתמונת ל"ט מהמאמר הראשון | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר קבצנו יהיה יחס ההפסד אל הריוח וההפסד מקובצים כיחס מה שמכר לערך המותיר אל כל המסחר | ||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
ולפי ששלשה מאלו ידועים לך תוכל להוציא הרביעי על האופן שקדם בראש זה השער | ||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון ראובן קנה ב' שביעיות המדה ג' די' ורביע די' והיה הממון ק' די' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה ערך המדה לפי מה שקנה י"א די' וג' שמיניות הדינר | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וערכה לפני הערך הראשון י"ד די' ורביע די' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ולפי הערך השני יהיה ערכה ט' די' וו' חלקים מכ"ה בדי' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ולזה יהיה המסחר ח' מדות וע"ב חלקים מצ"א בדי' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה מה שהרויח במדה לפי הערך הראשון הם ב' די' וז' שמיניות הדי' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ומה שהפסיד במדה לפי הערך השני הם ב' די' וכ"ז חלקים ממאתים בדי' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה ההפסד והריוח מקובצים הם ה' די' וב' חלקים ממאתים בדי' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ערוך סך דינרי ההפסד ושבריהם על סך מדות המסחר ושבריהן וחלק העולה על סך דינרי ההפסד והריוח מקובצים ושבריהם והעולה הם סך מדות המסחר שמכר לערך המותיר ושבריהם | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
ערכת ב' שלמים וכ"ח חלקים ממאתים באחד על ח' שלמים וע"ב חלקים מצ"א באחד וחלקת העולה על ה' שלמים וב' חלקים ממאתים באחד והעולה | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והנשאר מן המסחר מכר לערך המחסיר | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם אמר השואל שהרויח או הפסיד מספר מה מן הדי' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
תקח מספר המדות אשר ירויח בהם זה הסך אם הרויח או אשר יפסיד בהם זה הסך אם הפסיד ותוציאהו מן המסחר ושמרהו והנשאר מהמסחר תחלקהו על האופן הקודם ותמצא מה שמכר לערך המותיר ממנו | ||||||||||||||||||||||||||||||||||||||||||||||||
|
[806]והנשאר ממנו הוא מה שמכר לערך המחסיר | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם הפסיד תחבר מה שמכר לערך המחסיר עם השמור ואם הרויח תחברהו עם מה שמכר לערך המותיר | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם היתה השאלה שמכר כ' מדות לערך המותיר ורצינו לדעת כמה מן המדות מכר לערך המחסיר בדרך שלא ירויח ולא יפסיד | ||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה כיחס כ' מדות אל הנעלם כן יחס ב' די' וכ"ז חלקים ממאתים בדינר אל ב' די' וז' שמיניות הדינ' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ולזה תוכל לדעת הנעלם על האופן שקדם | ||||||||||||||||||||||||||||||||||||||||||||||||
Barter Problem |
|||||||||||||||||||||||||||||||||||||||||||||||||
|
וממה שקדם יתבאר לך אם החליף אדם מסחר מה במסחר אחר וערך החלקים מן המדה ממה שנתן מספר ככה מן הדינר | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה שתוציא ערך המדה לכלל אחד מהערכים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וכיחס ערך המדה שנתן אל ערך המדה שקבל כן יחס סך המדות שקבל אל סך המדות שנתן | ||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
ושלשה מאלו המספרים ידועים לך ולזה תוכל להוציא הרביעי | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן אם ידעת סך המדות שנתן ושקבל מקובצות הנה תוכל להוציא כמה מן המדות נתן וכמה קבל | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה שתוציא ערך המדה לכל אחד מהערכים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וכיחס ערך המדה ממה שנתן אל מקובץ ערכי המדה ממה שנתן ושקבל כן יחס סך המדות שקבל אל מקובץ סך המדות שנתן ושקבל | ||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה השלשה מאלו המספרים ידועים לך ומהם תוכל לדעת הרביעי | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן יתבאר לך אם החליף אדם מסחר מה בשני מסחרים וערך חלקים מה מן המדה ממה שנתן מספר ככה מן הדי' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה תוציא ערך מה שנתן בכללו ותחלקהו על מספר המדות שקבל והוא יהיה ערך המדה המעורבת משני אלו המסחרים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וכבר[810] קדמה לך הדרך כמה חלקים מהמדה ילקחו מהמסחר האחד וכמה[811] מן המסחר [812]השני ויהיה ערך המדה ככה | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ולזה תכפיל מספר חלקי המדה שיקח מהמספר האחד על מספר מדות שני המסחרים שקבל והחלקים ההם הם חלקי המדה שקבל מזה המסחר | ||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
והשאר קבל מהמסחר השני | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וסבת זה מבוארת עם מה שקדם מהדברים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון ראובן נתן לשמעון ז' מדות ממסחר מה ערך המדה ב' דינרין ושביעית וקבל משמעון ט' מדות משני מסחרים ערך המדה מהמסחר האחד דינר וחצי וערך המדה מהמסחר השני ב' די' וג' רביעיות | ||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה ידענו שערך מה שקבל הם ט"ו די' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ולזה יהיה ערך המדה ממה שקבל די' וב' שלישיות | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ולפי שהיה ערך המדה מהמסחר האחד די' וחצי ומהמסחר השני ב' די' וג' רביעיות[813] הנה יקח ב' חלקים מט"ו במדה מהמסחר אשר ערך המדה ממנו ב' די' וג' רביעיות | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והיה ערך המדה די' וב' שלישיות הדינר | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה יתבאר כשהשיבונו כל שברי הדינרין אל חלקים ממין אחד רצוני השליש והחצי והרביע וזה שחסרון המחסיר הוא שנים מאלו החלקים ויתרון המוסיף הוא י"ג מאלו החלקי' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ובהיות הענין כן הוא מבואר שהוא יקח מהמסחר אשר ערך מדה ממנו די' וחצי ז' מדות וד' חומשי המדה | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והשאר יקח מהמסחר האחר | ||||||||||||||||||||||||||||||||||||||||||||||||
Give and Take Problem | |||||||||||||||||||||||||||||||||||||||||||||||||
|
וכזה התבאר לך אם התנה אדם לתת בשכירות לאיש מה בזמן מה מספר ככה מהדי' ובכל יום שיבטל הפועל יתן לשוכר מספר ככה מהדי' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה שתוציא הסך מהדי' שירויח הפועל ביום כשיעשה מלאכתו והסך מהדי' שיפסיד ביום כשיבטל | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וכיחס סך דינרי ההפסד אל סך דינרי הריוח כן יחס הזמן שבטל אל הזמן שעשה וזה מבואר בנפשו | ||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
[814]והשלשה מאלו המספרים ידועים ומהם תוציא הרביעי | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן אם היה ידוע לך[815] מקובץ שני הזמנים תוכל לדעת באופן הקודם כמה מן הזמן בטל וכמה עשה | ||||||||||||||||||||||||||||||||||||||||||||||||
Barter Problem |
|||||||||||||||||||||||||||||||||||||||||||||||||
|
שאלה איש אחד החליף מסחר מה במסחר אחר וערך חלקים מה מן המדה ממה שנתן ככה | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והמשל שהמסחר שנתן ישוו ג' חמישיותיו המדה עם ב' תשיעיותיו ב' די' והמסחר שקבל ישוו ג' חלקים מי"א במדה עם ב' שביעיותיה ג' די' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
הדרך בזה שתקח המורה אל כל החלקים הן מהמדה הן מהדנרין אם קרה שיהיו שם חלקים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וממנו תקח החלקים שילקחו בדי' לכל אחד מאלו הערכים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וכמו חלקי הדי' ממה שנתן אל חלקי הדי' ממה שקבל כן יחס מה שנתן אל מה שקבל | ||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה המורה לכל אלו החלקים הוא ג' אלפים תס"ה | ||||||||||||||||||||||||||||||||||||||||||||||||
|
לקחנו ג' חמישיותיו וב' תשיעיותיו והנה אלפים תס"ד | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ולזה יהיו החלקים שילקחו בדינר ממה שנתן אלף רל"ב | ||||||||||||||||||||||||||||||||||||||||||||||||
|
לקחנו ב' שביעיותיו וג' חלקים מי"א במדה בו ועלה אלף תתקל"ה | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ולזה יהיו החלקים שילקחו בדי' ממה שקבל תרמ"ה | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה כיחס אלף רל"ב אל תרמ"ה כן יחס מה שקבל אל כ' מדות | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וכבר ידעת דרך לקיחת זה המספר הנעלם | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וסבת זה מבוארת | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והקש על זה | ||||||||||||||||||||||||||||||||||||||||||||||||
Give and Take Problems |
|||||||||||||||||||||||||||||||||||||||||||||||||
|
ומזה התבאר לך אם התנה אדם לתת בשכירות לאיש מה בזמן מה מספר ככה מהדינ' ובכל יום שיבטל הפועל יתן לשוכר מספר ככה מהדינ' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
משל זה שראובן שכר שמעון לעשות מלאכתו ויתן לו בשכירותו בז' ימים וחומש יום כ' די' ושמעון יתן לראובן בכל יום שיבטל ד' חלקים מי"א בדי' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
לקחנו המורה לכל החלקים בכללם ועלה שפ"ה והוא חלקי היום | ||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה נראה בכמה חלקים מאלו ירויח די' ובכמה חלקים מאלו יפסידהו וכיחס מספר חלקי הריוח אל מספר חלקי ההפסד כן יחס ב' ימים וג' שביעיות אל הזמן שבטל | ||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה בקל"ח חלקים וי"ב חלקים מעשרים בחלק ירויח די' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ובאלף ונ"ח חלקים וג' חלקים מארבעה בחלק יפסיד די' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וככה יחס ב' ימים וג' שביעיות אל הזמן שבטל | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והקש על זה | ||||||||||||||||||||||||||||||||||||||||||||||||
Find a Number Problems |
|||||||||||||||||||||||||||||||||||||||||||||||||
Two Numbers |
|||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם שאל לך אדם כמה המספר שיהיו חלקים מה ממנו כמו חלקים מונחים ממספר מונח | ||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה הדרך בזה התבארה בתמונת נ"א | ||||||||||||||||||||||||||||||||||||||||||||||||
|
[816]שאלה הכינו מספר במספר מה והיה העולה ככה ומקובץ שני המספרים ככה | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
קח מרובע חצי מקובץ שני המספרים וגרע ממנו העולה ומהנשאר הוצא השרש הרבועיי והוסיפהו על החצי ממקובץ[817] שני המספרים והוא המספר האחד | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם גרענו מהחצי יהיה לך המספר השני | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
והמשל שיהיו שני המספרים מקובצים י"ג ושטח זה בזה י"ז | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וידענו שמרובע חצי מספר י"ג[818] הוא מ"ב ורביע | ||||||||||||||||||||||||||||||||||||||||||||||||
|
גרענו ממנו י"ז ונשאר כ"ה ורביע | ||||||||||||||||||||||||||||||||||||||||||||||||
|
הוצאנו שרשם והנה ה' שלמים וראשון אחד כ"ט שניים מ"ו ל"ד | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
הוספנום על ו' וחצי שהוא חצי י"ג והנה יהיה המספר האחד י"א שלמים ל"א ראשונים כ"ט מ"ו ל"ד | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
והמספר השני הוא אחד שלם כ"ח ראשונים ל' י"ג כ"ו | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה שטח זה בזה הוא י"ז בקירוב גדול | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה אי אפשר המצא זה המספר בדיוק מפני שאין לכ"ה ורביע שרש אמתי לפי מה שהתבאר | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה שיחס כ"ה ורביע אל כ"ה הוא כיחס מאה ואחד אל מאה | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ואין יחס מאה ואחד אל מאה[819] כיחס מספר מרובע אל מספר מרובע | ||||||||||||||||||||||||||||||||||||||||||||||||
|
שאם היה הדבר כן היה מאה ואחד מרובע לפי שמאה הוא מרובע | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם היה מאה ואחד מרובע היה שרשו אחדים שלמים וזה שקר | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם שאל הכינו מספר מונח בחלק מונח ממנו וקבצנו העולה עם שטח החלק המונח בשארית המספר המונח והיה ככה כמה כל אחד מהחלקים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
קח מרובע כל המספר וגרע ממנו זה[820] המספר המקובץ מהכאת המספר בחלק אחד ממנו וחלק אחד ממנו באחר ומהנשאר הוצא השרש והוא החלק האחד והנשאר מהמספר הוא החלק המונח | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
והמשל שיהיה שטח עשרה בחלק מונח ממנו עם שטח החלק ההוא בחלק השני שמנים ורצינו לדעת כמה החלק המונח | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה מרובע עשרה הוא [821]מאה | ||||||||||||||||||||||||||||||||||||||||||||||||
|
גרענו מהם שמנים ונשארו עשרים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
הוצאנו שרשם והוא בדרך קירוב ד' שלמים כ"ח י"ט מ"א כ"א והוא החלק האחד | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
והנשאר שהוא ה' שלמים ל"א מ' י"ח ל"ט הוא החלק המונח | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תכהו בעשרה ובנשאר יעלה פ' בקירוב גדול | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם שאל הכינו מספר מונח בחלק מונח ממנו וקבצנו העולה עם מרובע החלק הנשאר והיה ככה ורצינו לדעת כמה כל אחד מהחלקים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
גרע הכל ממרובע כל המספר והנשאר בידך גרעהו ממרובע חצי המספר והנשאר בידך[822] הוצא את יסודו והוסיפהו על חצי המספר והנה החלק האחד והנשאר מהמספר הוא החלק השני | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
והמשל שיהיה שטח עשרה בחלק אחד ממנו עם מרובע הנשאר פ' ורצינו לדעת כמה כל אחד מהחלקים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
גרענו פ' ממאה ונשארו עשרים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
גרענו עשרים מכ"ה ונשארו חמשה | ||||||||||||||||||||||||||||||||||||||||||||||||
|
הוצאנו שרשם והוא בקירוב ב' שלמים י"ד ט' נ' מ' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
הוספנום על חמשה והנה החלק האחד ז' שלמים י"ד ט' נ' מ' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
והחלק השני ב' שלמים מ"ה נ' ט' כ' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה שטח י' באי זו חלק שיהיה מאלו עם מרובע החלק הנשאר הוא שמנים בקירוב | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והוא מבואר במעט עיון ממה שקדם שבאי זה מהחלקים שיוכה מספר עשרה ויחובר עם מרובע הנשאר יהיה העולה אחד בעינו | ||||||||||||||||||||||||||||||||||||||||||||||||
|
לפי שהעולה יהיה שוה לשטח החלק האחד בשני ולמרובעי שניהם | ||||||||||||||||||||||||||||||||||||||||||||||||
|
שאלה חברנו מספר מונח ראשון עם חלקים מונחים ממספר מונח שני והיה העולה שוה למספר המונח השני עם חלקים אחרים מהמספר המונח הראשון והיה העולה מספר ככה כמה כל אחד מהמספרים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
הדרך בזה שתשיב החלקים חלק | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ואחר כן תוציא המספרים שינהגו זה המנהג על האופן שהתבאר בתמונת נ"ב וזה יתבאר מהמופת ההוא בעינו | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן ראוי שתדע שכל מה שהתבאר בתמונת מ"ז ומ"ח ונ"ג יצדק בחלקים גם כן כשיושבו חלק וזה יתבאר גם כן מהמופת אשר באלו התמונות | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ואחר שישלם לך המעשה בהוצאת המספרים אשר ינהגו זה המנהג תדע מה יעלה [823]המספר האחד עם החלקים המונחים מהמספר השני | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והוא הגיליי לעולה הידוע ותוכל להוציא הגיליים לשני המספרים על האופן שהתבאר בראש זה השער | ||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה שיחובר המספר המונח הראשון עם ב' שביעיות ותשיעית מהמספר המונח השני והיה עשרים ואם יחובר המספר המונח השני[824] עם ב' חמישיות המספר המונח הראשון יהיו עשרים גם כן | ||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה נשיב ב' שביעיות ותשיעית חלק אחד והנה יהיה חלק אחד[825] משנים וי"ג חלקים מכ"ה באחד | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן נשיב הב' חמישיות חלק אחד ויהיו חלק אחד משנים וחצי | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ואחר כן נוציא שני מספרים ינהגו זה המנהג על הצד שהתבאר בתמונת נ"ב | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ולזה נערוך אחד וי"ג חלקים מכ"ה באחד על ב' שלמים וחצי ועלה ג' וד' חומשין והוא המספר הראשון | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ונערוך אחד וחצי על ב' וי"ג חלקים מכ"ה ויעלה ג' שלמים ול"ט חלקים מנ' והוא המספר השני | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה המספר הראשון עם ב' שביעיות המספר השני ותשיעיתו הוא ה' שלמים וג' עשיריות וככה המספר השני עם ב' חמישיות המספר הראשון | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ולפי שידעת הגיליי לה' שלמים וג' עשיריות והוא עשרים תוציא הגיליים למספר הראשון והשני על זה היחס והנה המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ערכת הראשון על עשרים וחלקת על ה' שלמים וג' עשיריות והנה המונח הראשון הוא י"ד שלמים וי"ח חלקים מנ"ג | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ובזה הדרך יהיה המונח השני י"ד שלמים וי"ד חלקים מנ"ג באחד | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והקש על זה | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||
|
והיה זה כן לפי שיחס הראשון אל המונח הראשון כיחס העולה אל עשרים וכיחס השני אל המונח השני | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וכזה הוא מבואר שיחס ב' חומשי הראשון על ב' חומשי המונח הראשון כיחס הראשון אל המונח הראשון | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וכזה יהיה יחס ב' שביעיות השני ותשיעיתו אל ב' שביעיות המונח השני ותשיעיתו כיחס | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר קבצנו שנים גיליים יהיה גם כן יחס מקובצם אל מקובץ [826]גיליהם כיחס העולה אל עשרים ולזה יהיה יחס השני וב' חומשי הראשון מקובצים אל המונח השני וב' חומשי המונח הראשון מקובצים כיחס העולה שהוא ה' שלמים וג' עשיריות אל עשרים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר המירונו הנה יהיה [יחס] השני וב' חומשי הראשון מקובצים אל העולה כיחס המונח השני וב' חומשי המונח הראשון אל עשרים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
אבל הראשון מאלו הארבעה שוה לשני | ||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה [ה]שלישי[827] ישוה לרביעי הנה אם כן המונח השני וב' חומשי המונח הראשון הוא עשרים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ובזה התבאר שהמונח הראשון וב' שביעיות המונח השני ותשיעיתו הוא עשרים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והיתה השאלה לחבר עם הראשון כפלים מה מהשני ועם השני כפלים מה מהראשון ויהיה העולה אחד בעינו | ||||||||||||||||||||||||||||||||||||||||||||||||
|
תוציא ממספר כפלי [ה]ראשון אחד והנשאר הוא השני וכזה תוציא [מ]מספר כפלי השני אחד והנשאר הוא הראשון | ||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון שיהיה הראשון עם שני כפלי השני [וחציו] כמו השני עם שלשה כפלי הראשון ורביעיתו | ||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה תשים הראשון אחד וחצי [והשני ב' שלמים ורביע | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ולזה יהיה הראשון עם שני כפלי השני וחציו] הוא הראשון והשני מקובצים ושטח אחד וחצי שהוא הראשון בשני שהוא ב' שלמים ורביע | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
וכזה יהיה השני עם שלשה כפלי הראשון ורביעיתו שוה לשני ולראשון מקובצים ולשטח השני שהוא שנים ורביע באחד וחצי שהוא הראשון | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
ולזה הוא מבואר שהעולה הוא אחד בעצמו והוא ז' שלמים ושמינית | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם אמר השואל שהראשון הוא עשרים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה תוכל להוציא כמה השני וזה שיחס הראשון אל עשרים כיחס השני שהוא ב' ורביע אל הנעלם | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ולזה יהיה השני שלשים | ||||||||||||||||||||||||||||||||||||||||||||||||
Three Numbers |
|||||||||||||||||||||||||||||||||||||||||||||||||
| This is explained in Propositions 48, and 53 for fractions and for unit fractions, in the proof itself there, by converting the fractions to a unit fraction. | והנה בתמונת מ"ח ונ"ג הדבר מבואר עם חלקים כמה שהוא עם חלק מהמופת אשר שם בעינו וזה כשיושבו החלקים חלק | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
| The same issue is also in proposition 47, where the first number was 2, after we converted the fractions to a unit fraction.
|
וכן הענין בתמונת מ"ז כשהיה הראשון שנים אחר שהשיבונו החלקים חלק | ||||||||||||||||||||||||||||||||||||||||||||||||
| But, the way to extract these numbers is not explained there for a number that is less than 2.
|
אבל אם היה פחות | ||||||||||||||||||||||||||||||||||||||||||||||||
| We say that it is a reverse case of the case where the first number is greater than 2, because, then the first of these numbers is the third plus the excess of the second over the first, when we subtract from it the product of two minus the first by the product of the second by the third. The second and the third are extracted according the way explained there.
|
ונאמר שהדבר באלו הפך הענין כשהיה הראשון [828]מוסיף על שנים וזה שאז היה הראשון מאלו המספרים השלישי ויתרון השני על הראשון כשחסרנו ממנו השטח ההווה מחסרון הראשון משנים בשטח השני בשלישי והשני והשלישי יוצאו על הצד שהתבאר שם | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והמשל שיהיה המספר אשר ממנו נקח חלק אחד כשהשיבונו החלקים חלק אחד פחות משנים והוא א' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ויהיה חסרונו משנים קצה אחד והיא ד' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ויהיה המספר השני ב' ויהיה יתרונו על א' מספר ה' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ויהיה המספר [השלישי][829] ג' ויהיה יתרונו על ב' מספר ז' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ויהיה המספר הנמשך לפני ב' מספר ח' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והנמשך לפני ג' מספר ט' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והנמשך לפני א' מספר ל' והוא יתרון א' על אחד | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ויהיו כ'ל' שוים לא' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ולזה יהיה כ' אחד | ||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה [נגרע][830] ממספרי ג'ה' מורכב ד'ב'ג' ונשים הנשאר מ' והוא יהיה המספר הראשון | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ונחבר עם מ' כפל שטח ה' בט' ויהיה העולה נ' והוא יהיה המספר השני | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ונחבר עם נ' כפל שטח ל' בז' ונשים העולה ס' והוא יהיה המספר השלישי | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ונבאר שבאלו המספרים הם המבוקשים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
המופת שהמספר הראשון והוא מ' שוה למספרי ג'ה' פחות מספר ד'ב'ג' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והמספר השני והוא נ' שוה למספרי ג'ה' פחות מורכב ד'ב'ג' ולכפל שטח ה' בט' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
[והמספר השלישי והוא ס' שוה למספרי ג'ה' פחות מורכב ד'ב'ג' ולכפל שטח ה' בט'] ולכפל שטח ל' בז' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
ונאמר שהחלק הנקרא בא' ממספרי נ'ס'[831] שוה לכפל שטח ח' בט' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
המופת שחצי מספרי נ'ס'[832] שוים למספרי ג'ה' ולכפל שטח ה' בט' ולשטח ל' בז' כשחוסר מכל זה מורכב ד'ב'ג' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
ונשים שטח ל' בח'ג' משותף | ||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה אם כן חצי מספרי נ'ס' שוים למספרי ג'ה' ולכפלי שטח ה' בט' ולשטח ל' בז' ולשטח ל' בח'ג' כשחוסר מכל זה מורכב ד'ב'ג' ושטח ל' בח'ג' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
אבל שטח ה' בט' עם שטח ל' בז' שוה לשטח ח' במספרי ה'ז' מקובצים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
לפי שהיו הנה שלשה מספרים ל'ח'ט' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ויתרון ח' על ל' ה' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ויתרון ט'[833] על ח' ז' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה מספרי ה'ז'ל' שוים למספר ט' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
אם כן שטח ח' במספרי ה'ז' עם שטח ח' בל' יהיה שוה [834]לשטח ח' בט' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ונגרע משטח ל' בח'ג' [שטח][835] ל' בח' וישאר לך שטח ל' בג' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ומספרי ג'ה' ושטח ה' בט' כשחוסר מכל זה מורכב ד'ב'ג' ושטח ל' בח'ג' ואומר שזה כלו שוה למורכב ל'ח'ט' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
המופת שאנחנו נחבר שטח ה' בט' עם ה' ויהיה שוה לשטח ה' בג' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד נחברהו עם ג' ויהיה שוה לשטח ה'כ' בג' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד נחברהו עם שטח ל' בג' ויהיה העולה שוה לשטח ה'כ'ל' בג' וזה שוה לשטח ב' בג' וזה שוה לשטח ד'ל' שהוא אחד במורכב ב'ג' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
ונחסר מזה מורכב ד'ב'ג' וישאר מורכב ל'ב'ג' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה מורכב ל'ח'ט' פחות ממורכב ל'ב'ג' מורכב ל'ח'ג' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה שמורכב ל'ח' בט' עם מורכב ל'ח' שוה למורכב ל'ח'ג' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ומורכב ל'ח'ג' שוה לשטח ח' [בל'ג'][836] | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר חברנו עם זה מורכב ל'ג' היה העולה שוה לשטח [ב'][837] בל'ג' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
אם כן כשחסרנו ממורכב ל'ב'ג' מורכב ל'ח'ג' שיהיה לנו לחסר מהמספר היה הנשאר מורכב ל'ח'ט' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
אם כן חצי מספרי נ'ס' שוה למורכב כ'ח'ט' שהוא שטח ח' בט' ולמורכב ל'ח'[ט'][838] וזה שוה לשטח כ'ל' במורכב ח'ט' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
אבל כ'ל' הוא א' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
אם כן חצי מספרי נ'ס' שוים למורכב א'ח'ט' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
אם כן שטח ח' בט' הוא חלק נקרא בא' מהחצי מספרי נ'ס' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ולזה יהיה כפל שטח ח' בט' חלק נקרא בא' ממספרי נ'ס' מקובצים ונשים העולה מספר ע' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וגם כן הנה חצי מספרי מ'[ס'] כששמנו שטח ל' בב' משותף שוים למספר ה'ג' ושטח ה' בט' ושטח ל' בז' ושטח ל' בב' פחות מורכב ד'ב'ג' ושטח ל' בב' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
ואומר שזה כלו שוה למורכב ל'ב'ט' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה ששטח ה' בט' עם ה' שוה לשטח ה' בג' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וכשחובר [עם] שטח ה' בג' ג' יהיה שוה לשטח ה'כ' בג' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה שטח[839] ל' בב' עם שטח ל' בז' שוה לשטח ל' בג' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
לפי שה'כ'ל' כמו ב' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
יהיה אם כן זה שוה לשטח ל'ד' במורכב ב'ג' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
לפי של'ד' הוא אחד | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר חסרנו מזה מורכב [ד'ב'ג' ישאר מורכב ל'ב'ג' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר חסרנו מזה מורכב] ל'ב' ישאר שטח ט' במורכב ל'ב' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה אם כן חצי מספרי [מ'][840]ס' שוים למורכב ב'ל'ט' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
אם כן שטח [ל'][841] בט' הוא חלק נק[רא] בב' מחצי [842]מספרי [מ'ס'][843] מקובצים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ולזה יהיה כפל שטח ל' בט' חלק נקרא בב' ממספרי [מ'ס'][844] מקובצים ונשים העולה מספר [פ'][845] | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וגם כן הנה חצי מספרי מ'נ' כששמנו שטח ל' בג' משותף שוה למספרי ה'ג' ולשטח ה' בט' פחות מורכב ד'ב'ג' ושטח ל' בג' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
ואומר שזה כלו שוה למורכב ל'ח'ג' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה ששטח ה' בט' עם מספרי ה'ג' שוה לשטח ה'כ' בג' כמו שהתבאר קודם | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר חברנו עם זה שטח ל' בג' [היה עולה שטח ה'כ'ל' בג' שהוא שוה לשטח ב' בג'] יהיה אם כן זה שוה לשטח ל'ד' במורכב ב'ג' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
לפי של'ד' הוא אחד | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר חסרנו מזה מורכב ד'ב'ג' ישאר מורכב ל'ב'ג' שהוא שטח ב' במורכב ל'ג' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר חסרנו מזה מורכב ל'ג' ישאר שטח ח' במורכב ל'ג' וזה שוה למורכב ל'ח'ג' שהוא שטח ג' במורכב ל'ח' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
אם כן שטח ל' בח' הוא חלק נקרא בג' מחצי מספרי מ'נ' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ולזה יהיה כפל שטח ל' בח' חלק נקרא בג' ממספרי מ'נ' מקובצים ונשים העולה מספר צ' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ואומר שמספרי מ'ע' שוים למספרי נ'פ' או למספרי [ס'צ'][846] | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה שמספרי מ'ע' שוים למספר מ' ולכפל שטח ח' בט' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ונ'פ' שוים למספר מ' ולכפל שטח ה' בט' ולכפל שטח ל' בט' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
אם כן מספרי נ'פ' שוים גם כן למספר מ' ולכפל שטח ח' בט' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וגם כן הנה מספרי [ס'צ'][847] שוים למ' ולכפל שטח ה' בט' ולכפל שטח ז' בל' ולכפל שטח ח' בל' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
אבל כפל שטח ז' בל' [עם כפל שטח ח' בל'] שוה לכפל שטח ז'ח' בל' וזה שוה לכפל שטח ט' בל' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר חובר עם זה כפל שטח ט' בה' היה העולה כפל שטח ט' בל'ה' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
ול'ה' כמו ח' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ולזה יהיה העולה שוה לכפל שטח ט' בח' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר חברנו עם זה מספר מ' היה העולה גם כן מספר מ' וכפל שטח ח' בט' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והוא מה שרצינו | ||||||||||||||||||||||||||||||||||||||||||||||||
| Examples | |||||||||||||||||||||||||||||||||||||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| We will give you examples for each of the methods when there are fractions there, so that you will become wise and understand. | ונתן לך דמיונים על זה בכל אחד מהאופנים כשיהיו שם חלקים למען תשכיל ותדע | ||||||||||||||||||||||||||||||||||||||||||||||||
|
נרצה שנמצא שלשה מספרים יהיו האחד עם ב' שביעיות הנשארים כמו האחר עם ב' חמישיות הנשארים וכמו האחר עם ג' חלקים מי"א בנשארים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
השיבונו ב' שביעיות חלק אחד [848]והיה חלק אחד מג' וחצי | ||||||||||||||||||||||||||||||||||||||||||||||||
|
השיבונו ב' חמישיות חלק אחד והיה חלק אחד מב' וחצי | ||||||||||||||||||||||||||||||||||||||||||||||||
|
השיבונו ג' חלקים מי"א חלק אחד והיה חלק אחד מג' וב' שלישים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
חברנו המספר הגדול עם יתרון האמצעי על הקטן וחברנו עם זה השטח ההווה מיתרון הקטן על שנים בשטח האמצעי בגדול ולזה יהיה המספר הראשון י"א שלמים וחלק מי"ב באחד | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
חברנו עם זה כפל שטח יתרון האמצעי על הקטן בגדול פחות אחד ויעלה י"ו שלמים וי"א חלקים מי"ב באחד והוא המספר השלישי | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
והיה הראשון עם ב' חמישיות הנשארים שהוא חלק אחד מב' וחצי כמו השני עם ב' שביעיות הנשארים וכמו השלישי עם ג'[849] חלקים מי"א בנשארים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון שני נרצה שנמצא שלשה מספרים יהיה האחד עם חצי הנשארים כמו האחר עם ב' חמישיות הנשארים וכמו האחר עם ג' חלקים מי"א בנשארים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר השיבונו החלקים חלק יהיו המספרים אשר אלו החלקים נקראים בהן שנים ושנים וחצי וג' וב' שלישיות | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ונשים המספר הראשון הגדול ויתרון האמצעי על הקטן מקובצים ולזה יהיה הראשון ד' שלמים וששית אחד | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ויהיה השני על הצד הקודם ו' שלמים וה'[850] ששיות | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ויהיה השלישי ח' שלמים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ויהיה הראשון עם חצי הנשארים כמו השני עם ב' חמישיות הנשארים וכמו השלישי עם ג' חלקים בי"א בנשארים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון שלישי שנרצה שנמצא שלשה מספרים יהיה האחד עם ג' חמשיות הנשארים כמו האחר עם ד' חלקים מי"א בנשארים וכמו האחר עם ב' שביעיות הנשארים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר השיבונו אלו החלקים חלק יהיה הקטן מהמספרים אשר אלו החלקים נקראים בהם אחד וב' שלישיות והאמצעי ב' וג' רביעיות והגדול ג' וחצי | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה יהיה המספר הראשון על האופן שקדם אחד וט' חלקים מכ"ד | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ויהיה המספר השני ח' וב' חלקים מכ"ד | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והמספר השלישי הוא ט' וב' חלקים מכ"ד | ||||||||||||||||||||||||||||||||||||||||||||||||
Four Numbers |
|||||||||||||||||||||||||||||||||||||||||||||||||
| Another easy method to find as many numbers as there may be, one of which with a part or parts of the rest are the same as another with a part or parts of the rest. | דרך אחרת קלה למצוא מספרים כמה שיהיו יהיה האחד [851]עם חלק או חלקים מהנשארים [כמו האחר עם חלק או חלקים מהנשארים] | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והמשל שנרצה למצוא [ארבעה] מספרים יהיה האחד עם א' חלקים מד' מהנשארים כמו השני עם ב' חלקים מה' בנשארים וכמו השלישי[852] עם חלק אחד מז' בנשארים וכמו הרביעי עם ג' חלקים מ[ח'][853] בנשארים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה נשים א'ט' שוים למספר ד' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וב'כ' שוים למספר ה' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ול' פחות אחד מז' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וג'מ' שוים לח' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה נכה ד' במורכב כ'ל'מ' ונשים העולה נ' והוא יהיה מספר מקובץ שלשה המספרים מלבד הראשון | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וגם כן הנה נכה ה' במורכב ט'ל'מ' ונשים העולה ס' והוא יהיה מספר מקובץ שלשה המספרים מלבד [השני][854] | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וגם כן הנה נכה ז' [במורכב ט'כ'מ' ונשים העולה ע' והוא יהיה מספר מקובץ שלשה מספרים מלבד השלישי | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וגם כן הנה נכה ח'] במורכב ט'כ'ל' ונשים העולה פ' והוא יהיה מספר מקובץ שלשה המספרים מלבד הרביעי | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ואחר שידעת מקובץ כל שלשה מספרים מאלו הארבעה תוכל להוציא כל אחד מהם איש על מקומו | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והדרך היותר נאותה בזה שתקח המספר היותר קטן ממספרי נ'ס'ע'פ' ואחריו הקטון מהנשאר וכן עד שתגיע אל היותר גדול | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ונניח שיהיה היותר קטן מספר ע' והיותר קטן אחריו מספר נ' והיותר קטן אחריו מספר פ' ולזה יהיה יותר גדול ממספר ס' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ויהיה יתרון ס' על פ' מספר צ' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ויתרון ס' על נ' מספר ק' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ויתרון ס' על ע' מספר ר' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה מפני שס' הוא מקובץ הראשון והשלישי והרביעי ופ' הוא מקובץ הראשון והשני והשלישי הנה כאשר חסרנו מהם הראשון והשלישי משותפים נשאר יתרון הרביעי על השני הוא יתרון ס' על פ' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ולזה יהיה יתרון הרביעי על השני מספר צ' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וגם כן הנה מפני שמספר ס' הוא מקובץ הראשון והשלישי והרביעי ומספר נ' הוא מקובץ השני והשלישי והרביעי הנה כאשר חסרנו מהם השלישי והרביעי משותפים נשאר יתרון הראשון על השני מספר ק' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
וכזה יתבאר שיתרון השלישי על השני הוא מספר ר' לפי שיתרון ס' על ע' הוא מספר [ר'][855] | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ובהיות הענין כן הנה המספר השני הוא היותר קטן | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ונקח אחד ממקובץ המספרים אשר המספר השני בכללם [856]והוא ע' במשלינו זה | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ולפי שמקובץ ע' הוא המספר הראשון והשני והרביעי הוא מבואר שיתרון ע' על שלשה דמיוני השני הוא שוה ליתרון הראשון על השני וליתרון הרביעי על השני מקובצים והם מספרי ק'צ' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
ולזה נגרע ממספר ע' מספרי ק'צ' ונחלק הנשאר על מספר המספרים אשר מספר ע' מקובצם ר"ל על שלשה והעולה מן החלוקה הוא המספר השני ונניח שיהיה ש' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ולזה יהיה המספר הראשון ש'ק' מקובצים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והמספר השלישי מספרי ש'ר' מקובצים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והמספר הרביעי מספרי ש'צ' מקובצים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ואחר שמצאנו אלה המספרים הנה נבאר שאלו הם המספרים שינהגו זה המנהג הדרוש | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה שאנחנו נבאר שהנשאר מהמספרים האלו הוא תמיד שוה למורכב ט'כ'ל'מ' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
המופת שהראשון כשחובר עם א' חלקים מד' בנשארים היה הנשאר שטח ט' במורכב כ'ל'מ' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה שהנשארים שוים למספר נ' והוא שטח ד' במורכב כ'ל'מ' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה מספר נ' מדמיוני מורכב כ'ל'מ' מה שבמספר ד' מן האחדים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה מורכב כ'ל'מ' הוא חלק נקרא בד' ממספר נ' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ולזה יהיה בא' חלקים מד' במספר נ' מדמיוני מורכב כ'ל'מ' כמו מה שבמספר א' מן האחדים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ולזה יהיה בנשאר מאלו השלשה [המספרים][857] מדמיוני מורכב [כ'ל'מ'] כמו מה שבמספר ט' מן האחדים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
לפי שמספרי ט'א' שוים למספר ד' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה שוה למורכב ט'כ'ל'מ' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
וכזה התבאר שהשני כשחובר עם ב' חלקים מה' בנשארים היה הנשאר שוה לשטח כ' במורכב ט'ל'מ' וזה שוה למורכב ט'כ'ל'מ' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
וכזה יתבאר שהשלישי כשחובר עם חלק מז' מהנשארים היה הנשאר שוה לשטח ל' במורכב ט'כ'מ' וזה של' פחות אחד מז' וזה שוה למורכב ט'כ'ל'מ' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
ולפי שהנשאר מהארבעה מספרים הוא תמיד כמו מורכב ט'כ'ל'מ' יהיה בהכרח הלקוח מהם אחד בעינו | ||||||||||||||||||||||||||||||||||||||||||||||||
|
כי כשחוסרו מהשוים שוים יהיו הנשארים שוים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והוא מה שרצינו לבאר | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ואומר שאם היה מקובץ ק'[צ]'[858] [859]במשלינו זה יותר ממספר ע'[860] או שוה לו שהשאלה כוזבת בהכרח | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ר"ל שאי אפשר שימצאו מספרים ינהגו זה המנהג | ||||||||||||||||||||||||||||||||||||||||||||||||
|
המופת שאם היה אפשר הנה יהיו המספרים ההם מספרי ת'ך'ץ'[861]ף' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ויהיה ת' עם א' חלקים מד' במספרי ך'ץ'[862]ף' כמו ך' עם ב' חלקים מה' במספרי ת'ץ'[863]ף' או כמו ץ'[864] עם חלק אחד מז' במספרי ת'ך'ף'[865] או כמו ף'[866] עם ג' חלקים מח' במספרי ת'ך'ץ' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
ולזה יהיה הנשאר מהארבעה המספרים אחד בעינו והוא מספר ן' על דרך משל | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
ויתבאר שיחס ן' אל מספרי ך'ץ'[867]ף' מקובצים הוא כיחס ט' אל ד' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה שכאשר לקחנו מספרי ך'ץ'[868]ף' א'[869] חלקים מד' היה הנשאר מהם והוא ן' ט' חלקים מד' במספרי ך'ץ'[870]ף' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה שמספר א'ט' שוים למספר ד' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וכזה התבאר שיחס מספרי ת'ץ'ף' אל ן' כיחס ה' אל כ' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וכזה התבאר שיחס ת'ך'ף' אל ן' כיחס ז' אל ל' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וכזה [התבאר] שיחס ת'ך'ץ' אל ן' הוא כיחס ח' אל מ' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה נחלק מספר ן' בדמיוני מה שבמורכב ט'כ'ל'מ' מן האחדים ויהיה אחד ממורכב ט'כ'ל'מ' במספר ן' מספר ם' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה מפני שיחס ן' אל מספרי ת'ך'ף' הוא כיחס ל' אל ז' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ובמספר ן' מדמיוני ם' כמו מה שבשטח ל' במורכב ט'כ'מ' מן האחדים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה יהיה במספרי ת'ך'ף' מדמיוני ם' כמו מה שבמספר שטח ז' במורכב ט'כ'מ' מן האחדים ולזה יהיה במספרי ת'ך'ף' מדמיוני ם' כמו מה שבמספר ע' מן האחדים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ובזה התבאר שבמספרי ך'ץ'ף' מדמיוני ם' כמו מה שבמספר נ' מן האחדים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ושבמספרי ת'ץ'ף' מדמיוני ם' כמו מה שבמספר ס' מן האחדים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ושבמספרי ת'ך'ץ' מדמיוני ם' כמו מה שבמספר פ' מן האחדים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ולזה יתבאר בכמו הבאור הקודם שיתרון הראשון על השני הוא מדמיוני ם' כמו מה שבמספר ק' מן האחדים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ויתרון הרביעי על השני הוא מדמיוני ם' כמו מה שבמספר צ' מן האחדים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
|||||||||||||||||||||||||||||||||||||||||||||||||
|
ולזה יחוייב שיהיה ביתרון ך'ף' על שני דמיוני ת' מדמיוני מספר ם' כמו מה שבמספר ק'צ' מן האחדים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ונניח שיהיה במספר ת' מדמיוני ם' כמו מה שבמספר ו' מן האחדים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וכבר היה במספר ת'ך'ף' מדמיוני ם' כמו מה שבמספרי ק'צ' מן האחדים ושלשה דמיוני מה שבת' מדמיוני ם' אם כן במספרי ת'ך'ף' מדמיוני ם' כמו מה שבמספרי ק'צ' ושלשה דמיוני מספר ו' מן האחדים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
אבל במספרי ת'ך'ף' מדמיוני ם' כמו מה שבמספר ע' מן האחדים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
אם כן מספר ע' שוה למספרי ק'צ' ושלשה דמיוני מספר ו' מן האחדים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וכבר הנחנו שמספרי ק'צ' שוים למספר ע' או מוסיפים על מספר ע' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
זה שקר ר"ל שיהיה החלק שוה לכל או יותר מהכל | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ובהיות הענין כן הנה השאלה כוזבת | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והוא מה שרצינו לבאר | ||||||||||||||||||||||||||||||||||||||||||||||||
Seven Numbers |
|||||||||||||||||||||||||||||||||||||||||||||||||
| We give you an example of this: | והנה נתן לך דמיון על זה | ||||||||||||||||||||||||||||||||||||||||||||||||
|
נרצה שנמצא שבעה מספרים יהיה הראשון עם ב' שביעיות הנשאר כמו השני עם שליש הנשאר וכמו השלישי עם ב' תשיעיות הנשאר וכמו הרביעי עם ג' שמיניות הנשאר וכמו החמישי עם ששית הנשאר וכמו הששי עם רביעית הנשאר וכמו השביעי עם ב' חלקים מי"א חלקים בנשאר | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה לפי הבאור הקודם נכה ז' במורכב ב'ז'ה'ה'ג'ט' כמו שתראה בזאת הצורה והנה העולה ס"ו אלפים וק"נ והם השני והשלישי והרביעי והחמישי והששי והשביעי מקובצים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
הכינו ג' במורכב ה'ז'ה'ה'ג'ט' והנה העולה ע' אלפים ותתע"ה והם הא'ג'ד'ה'ו'ז' מקובצים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
הכינו ט' במורכב ה'ב'ה'ה'ג'ט' ועלה ס' אלפים תש"נ והם א'ב'ד'ה'ו'ז' מקובצים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
הכינו ח' במורכב ה'ב'ז'ה'ג'ט' ועלה ע"ה אלפים ות"ר והם א'ב'ג'ה'ו'ז' מקובצים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
הכינו ו' במורכב ה'ב'ז'ה'ג'ט' ועלה נ"ו אלפים וז' מאות והם א'ב'ג'ד'ו'ז' מקובצים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
הכינו ד' במורכב ה'ב'ז'ה'ה'ט' ועלה ס"ג אלפים והם הראשון והשני והשלישי והרביעי והחמישי והשישי והשביעי מקובצים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
הכינו י"א במורכב ה'ב'ז'ה'ה'ג' ועלה ע"ז אלפים תש"נ והם הא'ב'ג'ד'ה'ו' מקובצים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה היותר קטן מאלו המקובצים הוא נ"ו אלפים וז' מאות ואחריו ס' אלפים תש"נ ואחריו ס"ג אלפים ואחריו ס"ו אלפים וק"נ ואחריו ע' אלפים תתע"ה והאחריו ע"ה אלפים ת"ר ואחריו ע"ז אלפים תש"נ | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה יתרון ע"ז אלפים תש"נ על שלפניו הוא ו' אלפים ק"נ וככה יתרון הרביעי על השביעי | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ויתרונו על ע' אלפים ותתע"ה ו' אלפים תתע"ה וככה יתרון השני על השביעי | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ויתרונו על ס"ו אלפים וק"נ י"א אלפים ות"ר וככה יתרון הראשון על השביעי | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ויתרונו על ס"ג אלפים י"ד אלפים תש"נ וככה יתרון הששי על השביעי | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ויתרונו על ס' אלפים תש"נ י"ז אלפים תש"נ וככה יתרון השלישי על השביעי | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ויתרונו על נ"ו אלפים וז' מאות כ"א אלפים ונ' וככה יתרון החמישי על השביעי | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ולזה יהיה החמישי היותר גדול | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה מקובץ כל המספרים מלבד החמישי הוא נ"ו אלפים וז' מאות | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ויתרוני הראשון והשני והשלישי והרביעי והששי על חמשה דמיוני השביעי הוא נ"ג אלפים וקכ"ה | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
גרענו זה מנ"ו אלפים וז' מאות ונשאר ג' אלפים ותקע"ה | ||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקנו הנשאר על ששה שהוא מספר אלו המספרים אשר היה נ"ו אלפים וז' מאות מקובצים ועלה תקצ"ה שלמים וה' ששיות אחד וככה המספר השביעי | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
הוספנו על השביעי יתרון הששי עליו שהוא י"ד אלפים תש"נ והנה הששי והוא ט"ו אלפים שמ"ה וה' ששיות אחד | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
ולזה יהיה החמישי כ"א אלפים תרמ"ה וה' ששיות | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והרביעי אלפים תשמ"ה וה' ששיות | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והשלישי י"ח אלפים שמ"ה שלמים וה' ששיות אחד | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והשני ז' אלפים ת"ע וה' ששיות אחד | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והראשון י"ב אלפים קצ"ה וה' ששיות אחד | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תרצה תוכל לבחון זה | ||||||||||||||||||||||||||||||||||||||||||||||||
Four Numbers |
|||||||||||||||||||||||||||||||||||||||||||||||||
| If the problem is to take multiples of the rest so that the results are all one and the same | ואם היתה השאלה לקחת הכפלים מה מהנשארים ויהיה העולה אחד בעינו | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והמשל שנרצה למצא ארבעה מספרים יהיה הראשון עם כפלי א' מהנשארים כמו השני עם כפלי ב' מהנשארים וכמו השלישי עם כפלי ג' מהנשארים וכמו הרביעי עם כפלי ד' מהנשארים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה נקח המספרים הנמשכים לפני א'ב'ג'ד' והם ה'ז'ח'ט' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
רצוני שה' נמשך לפני א' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וז' לפני ב' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וח' לפני ג' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וט' לפני ד' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה נשים מורכב ז'ח'ט' מקובץ כל המספרים מלבד הראשון | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ומורכב ה'ח'ט' מקובץ כל המספרים מלבד השני | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ומורכב ה'ז'ט' מקובץ כל המספרים מלבד השלישי | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ומורכב ה'ז'ח' מקובץ כל המספרים מלבד הרביעי | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ואחר שידעת כל זה הנה תוציא כל המספרים איש על מקומו על האופן שקדם | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ונמצא שאלו המספרים הם המספרים המבוקשים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
המופת שהראשון כאשר חובר עם כפלי א' מהנשארים הנה העולה שוה לראשון ולדמיוני מורכב ז'ח'ט' כמו מה שבמספר א' מן האחדים וזה שוה לראשון ולנשארים ולדמיוני מורכב ז'ח'ט' כמו מה שבמספר א' פחות אחד מן האחדים וזה שוה למורכב ה'ז'ח'ט' אם כן העולה שוה לארבעה המספרים ולמורכב ה'ז'ח'ט' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
וכזה התבאר שהשני כאשר חובר עם כפלי ב' מהנשארים היה העולה שוה לארבעה המספרים ולמורכב ה'ז'ח'ט' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
והשלישי כאשר חובר עם כפלי ג' מהנשארים היה העולה שוה למקובץ כל המספרים ולמורכב ה'ז'ח'ט' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
ושהרביעי כאשר חובר עם כפלי ד' מהנשארים היה העולה שוה למקובץ כל המספרים ולמורכב ה'ז'ח'ט' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
והוא מה שרצינו לבאר | ||||||||||||||||||||||||||||||||||||||||||||||||
| We say that if the excess of the two numbers over twice the smallest is greater than the sum of the three or equal to it, then the question is a false. | ונאמר שאם היו יתרוני שני המספרים במשלינו זה על שני דמיוני הקטן יותר ממקובץ שלשתם או שוה לו שהשאלה כוזבת | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והמשל שיהיה הרביעי הוא היותר קטן | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ויהיה מורכב ה'ח'ט' מספר כ' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ויהיו יתרוני הראשון והשלישי על שני דמיוני הרביעי מספר ל' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ויהיה מספר ל' שוה למספר כ' או יותר גדול ממנו ואומר שהשאלה כוזבת ושאי אפשר שימצאו מספרים ינהגו זה המנהג | ||||||||||||||||||||||||||||||||||||||||||||||||
|
המופת שאי אפשר שאם היה אפשר יהיו המספרים ההם מספרי מ'נ'ס'ע' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה נחלק מקובץ מ'ס'ע' בדמיון מה שבמורכב ה'ח'ט' מן האחדים ויהיו חלקיו על זה האופן שוים למספר פ' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה במקובץ מ'ס'ע' מדמיוני פ' כמו מה שבמורכב ה'ח'ט' מן האחדים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר חברנו עם ב' מכפלי מ'ס'ע' כמו מה שבמספר ז' מן האחדים לפי שמספר ז' הוא פחות אחד מב' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
אבל במ'ס'ע' מדמיוני פ' כמו מה שבמורכב ה'ז'ח'ט' מן האחדים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ולפי שהיה מ' מכפלי א' מהנשארים כמו נ' עם כפלי ב' מהנשארים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והיה מ' עם כפלי א' מן הנשארים שוה למספרי מ'נ'ס'ע' ולדמיוני נ'ס'ע' כמו מה שמספר ה' מן האחדים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
אם כן ה' מוכה על נ'ס'ע' יהיו בו מדמיוני פ' כמו מה שבמורכב ה'ז'ח'ט' מן האחדים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ולזה יהיה במורכב נ'ס'ע' מדמיוני פ' כמו מה שבמורכב ז'ח'ט' מן האחדים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ובזה יתבאר שבמספרי מ'נ'ע' מדמיוני פ' כמו מה שבמורכב ה'ז'ט' מן האחדים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ושבמספרי מ'נ'ס' מדמיוני פ' כמו מה שבמורכב ה'ז'ח' מן האחדים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ולזה יהיו במספרי מ'ס'ע' מדמיוני פ' כמו מה שבמספר כ' מן האחדים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ויתבאר בכמו הביאור הקודם שיתרון מ'ס' על שני דמיוני ע' מדמיוני פ' כמו מה שבמספר ל' מהאחדים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
אבל ל' כמו כ' או יותר | ||||||||||||||||||||||||||||||||||||||||||||||||
|
אם כן מספרי מ'ס' יותר גדולים ממספרי מ'ס'ע' וזה שקר | ||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה אם כן אי אפשר שימצאו מספרים ינהגו זה המנהג | ||||||||||||||||||||||||||||||||||||||||||||||||
Five Numbers |
|||||||||||||||||||||||||||||||||||||||||||||||||
| We will give you an example of this: | והנה נתן לך דמיון על זה | ||||||||||||||||||||||||||||||||||||||||||||||||
|
נרצה שנמצא חמשה מספרים יהיה הראשון עם שלושה דמיוני הנשארים כמו השני עם שלשה דמיוני הנשארים וחצי וכמו השלישי עם שלשה דמיוני הנשארים ושלישיותם וכמו הרביעי עם שלשה דמיוני הנשארים וב' שלישיותיהם וכמו החמישי עם ארבעה דמיוני הנשארים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה יהיה מקובץ הנשארים מלבד הראשון על האופן הקודם מ"ו וב' שלישיות | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ומקובץ הנשארים מלבד השני ל"ז ושליש | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ומקובץ הנשארים מלבד השלישי מ' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ומקובץ הנשארים מלבד הרביעי ל"ה | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ומקובץ הנשארים מלבד החמישי ל"א ותשיעית | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה יתרון השלישי על הראשון ו' וב' שלישיות | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ויתרון השני על הראשון ט' ושליש | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ויתרון הרביעי על הראשון י"א וב' שלישיות | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ויתרון החמישי על הראשון ט"ו וה' תשיעיות | ||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה אם כן יתרוני השני והשלישי והרביעי על שלשה דמיוני הראשון הוא כ"ז וב' שלישיות | ||||||||||||||||||||||||||||||||||||||||||||||||
|
גרענו מזה מקובץ ארבעתם שהוא ל"א ותשיעית ונשאר ג' וד' תשיעיות | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקנום על מספר אלו המספרים והנה הראשון ל"א חלקים מל"ו באחד | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והשני י' וז' חלקים מל"ו | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והשלישי ז' וי"ט חלקים מל"ו | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והרביעי י"ב וי"ט חלקים מל"ו | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והחמישי י"ו וט"ו חלקים מל"ו | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תרצה תוכל לבחון זה | ||||||||||||||||||||||||||||||||||||||||||||||||
Three Numbers |
|||||||||||||||||||||||||||||||||||||||||||||||||
|
שאלה חברנו מספר ראשון עם מספר שני והיה יחס העולה אל מספר שלישי מספר מונח וכשחברנו המספר הראשון אל המספר [873]השלישי היה יחס העולה אל המספר השני מספר מונח שני | ||||||||||||||||||||||||||||||||||||||||||||||||
|
כבר ידעת איך תמצא שלשה מספרים יצדק בהם זה ותוציאם | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ולפי שידעת אחד מן הגיליים לאחד מהם תוכל להוציא הגיליים למספרים הנשארים והנה המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון שיהיה מספר ראשון כשחובר אל מספר שני היה יחסו אל השלישי ג' שלמים וב' חמשיות ושביעית וכשחובר הראשון אל השלישי יהיה יחסו אל השני[874] ז' שלמים וב' שלישיות ורביעית | ||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה נוציא תחלה ג' מספרים ינהגו זה המנהג על זה הדרך שהתבאר במאמר הראשון מזה הספר | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ולזה תגרע אחד משטח ג' שלמים וב' חמישיות ושביעית בז' שלמים וב' שלישיות ורביעית וישאר כ"ז שלמים ושלישית שביעית והוא המספר הראשון | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ותוסיף אחד על ג' שלמים וב' חמישיות ושביעית ויהיו בידך ד' שלמים וב' חמישיות ושביעית[875] והוא המספר השני | ||||||||||||||||||||||||||||||||||||||||||||||||
|
גם תוסיף אחד על ז' שלמים וב' שלישיות ורביעית והעולה בידך הוא המספר השלישי והוא ח' שלמים וב' שלישיות ורביעית | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וכבר ידעת שהמספר הגיליי לשני הוא שלשים | ||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
|||||||||||||||||||||||||||||||||||||||||||||||||
|
ולזה יהיה המספר הגיליי לראשון קע"ח שלמים וצ"ח חלקים מקנ"ט באחד | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והמספר השלישי נ"ח שלמים ורפ"א חלקים משי"ח באחד | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ואלו השלשה מספרים הם [ה]מבוקשים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ואלו תחקור [ו]תמצא | ||||||||||||||||||||||||||||||||||||||||||||||||
| It has already been explained to you, if you know the sum of two of these successive numbers, how you can know all the numbers each corresponding to the known proportional number. | וכבר התבאר לך שאם ידעת מקובץ שנים מאלו הנמשכים איך תוכל לדעת כל המספרים איש על מקומו הגיליים לראשונים הידועים | ||||||||||||||||||||||||||||||||||||||||||||||||
| Or, if you know the excess of one of the successive proportional numbers, or of the sum of two of the successive proportional numbers, over another one of them, or over the sum of two of the successive proportional numbers. | או אם ידעת יתרון אחד מהנמשכים ידוע הגיליות או מקובץ שנים מהם ידועי הגיליות על אחד מהם ידוע הגיליות או על מקובץ שנים מהם ידועי הגיליות | ||||||||||||||||||||||||||||||||||||||||||||||||
| Or, other methods by which you can find the proportional numbers, each corresponding to its place. | ושאר האופנים אשר תוכל לקנות מהם ידיעת המספרים הגיליים איש על מקומו | ||||||||||||||||||||||||||||||||||||||||||||||||
| After you know these proportional numbers by whatever method you acquire this knowledge, we explain to you that these proportional numbers are the required numbers: | ואחר שתדע [876][המספרים] הגיליים מאיזה צד שתקנה הידיעה בזה הנה נבאר לך שאלו [המ]ספר[ים] הגיליים הם המבוקשים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה לפי שיחס הראשון מהקודמים אל השני מהם כיחס הראשון מהנמשכים אל השני מהם | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ויחס השני מהקודמים אל השלישי מהם כיחס השני מהנמשכים אל השלישי מהם | ||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה ביחס השווי יהיה יחס הראשון מהקודמים אל השלישי כיחס הראשון מהנמשכים אל השלישי | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר קבצנו הנה יחס הראשון והשני מהקודמים מקובצים אל השלישי כיחס הראשון והשני מהנמשכים מקובצים אל השלישי | ||||||||||||||||||||||||||||||||||||||||||||||||
|
אבל יחס הראשון והשני מהקודמים מקובצים אל השלישי הוא במשלינו זה ג' שלמים וב' חמישיות ושביעית | ||||||||||||||||||||||||||||||||||||||||||||||||
|
אם כן יחס הראשון והשני מהנמשכים מקובצים אל השלישי הוא ג' שלמים וב' חמישיות ושביעית | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וכזה גם כן התבאר שיחס הראשון והשלישי מהנמשכים מקובצים אל השני הוא ז' שלמים וב' שלישיות ורביעית | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והקש על זה | ||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון אחר יהיה יחס הראשון והשני מקובצים אל השלישי ג' חמשיות וששית ויחס הראשון והשלישי מקובצים אל השני ב' שלמים ושלישית | ||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה לפי מה[877] שהתבאר נוציא המספרים אשר מנהגם זה המנהג | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ויהיה המספר הראשון לפי מה שקדם ב' חמישיות ושלישית ושליש ששית | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ויהיה המספר השני אחד שלם וג' חמישיות וששית | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ויהיה מספר השלישי ג' שלמים ושלישית | ||||||||||||||||||||||||||||||||||||||||||||||||
|
|||||||||||||||||||||||||||||||||||||||||||||||||
|
ולפי שהיה המספר הגיליי לראשון עשרים יהיה המספר הגיליי לשני מ"ד שלמים ונ"ו חלקים מע"א באחד שלם | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והמספר הגיליי לשלישי פ"ד שלמים ול"ו חלקים מע"א באחד שלם | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והם המספרים המבוקשים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תרצה תוכל לבחון זה | ||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||
| You should know that if the product of the ratio of the first and the second summed together to the third by the ratio of the first and the third summed together to the second is not greater than one whole than whoever asked made a mistake.
|
וראוי שתדע שאם לא עלה יותר מאחד שלם השטח ההווה מיחס הראשון והשני מקובצים אל השלישי ביחס הראשון והשלישי מקובצים אל השני שכבר טעה השואל | ||||||||||||||||||||||||||||||||||||||||||||||||
| We offer a proposition to explain this. We explain in it that for any two given numbers, the product of the ratio of the first to the second by the ratio of the second to the first is one whole.
|
ונציע לביאור זה הקדמה נבאר בה [878]שכל שני מספרים מונחים הנה שטח יחס הראשון אל השני ביחס השני אל הראשון שלם | ||||||||||||||||||||||||||||||||||||||||||||||||
| This is because one of the given numbers is either one part or of the other, or parts [of it]. | וזה שאחד מהמספרים המונחים אם שיהיה חלק מהאחר או חלקים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ויהיה תחלה חלק ונאמר שכבר יתאמת מה שאמרנו | ||||||||||||||||||||||||||||||||||||||||||||||||
|
המשל שיהיו המספרים המונחים מספרי א'ב' והיה ב' ימנהו א' בשעור אחדי ג' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
אם כן יחס ב' אל א' מספר ג' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ויחס א' אל ב' חלק אחד מג' חלקים באחד | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר הוכה ג' שלמים על חלק אחד מג' חלקים באחד היה העולה אחד לפי מה שהתבאר קודם | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ויהיה גם כן המספר הקטן חלקים מן הגדול | ||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה אומר ששטח יחס המספר האחד אל האחר ביחס המספר האחר אליו הוא אחד | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ויהיה במשלינו זה המספר הקטן ד'ה' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והמספר הגדול ב' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ויהיה ד'ה' ז' חלקים מג' במספר ב' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ויהיה ה'ח' חלק מג' במספר ב' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה ה'ח' הוכה בז' והיה ה'ד' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ונכה ב' בז' ויהיה ט'כ' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה יחס ח'ה' אל ב' כיחס ד'ה' אל ט'כ' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר המירונו וחלפנו הנה יחס ד'ה' [אל ח'ה' כיחס ט'כ' אל ב' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וגם כן הנה יחס ד'ה'] אל ב' הוא ז' דמיוני יחס ח'ה' אל ב' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ויחס ב' אל ד'ה' הוא חלק אחד מז' מיחס ט'כ' אל ד'ה' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
אבל יחס ט'כ' אל ד'ה' שהוא ג' כבר יוכה ביחס ח'ה' אל ב' שהוא כיחס ד'ה' אל ט'כ' ויהיה אחד | ||||||||||||||||||||||||||||||||||||||||||||||||
|
אם כן יחס ד'ה' אל ב' כבר יוכה ביחס ב' אל ד'ה' ויהיה אחד גם כן לפי שהצלעות מספיקות | ||||||||||||||||||||||||||||||||||||||||||||||||
| When this is clarified, it is clear that the product of the ratio of the first and the second summed together to the third by the ratio of the first and the third summed together to the second is greater than one.
|
וכאשר התבאר זה הנה יתבאר שהשטח ההווה מיחס הראשון והשני מקובצים אל השלישי ביחס הראשון והשלישי מקובצים אל השני הוא יותר מאחד שלם | ||||||||||||||||||||||||||||||||||||||||||||||||
|
[879][וזה שיחס השלישי והראשון מקובצים אל השני הוא יותר גדול הרבה מיחס השלישי אל הראשון והשני מקובצים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ואולם שטח יחס הראשון והשני מקובצים אל השלישי ביחס השלישי אל הראשון והשני מקובצים הוא אחד שלם | ||||||||||||||||||||||||||||||||||||||||||||||||
|
אם כן השטח ההווה מיחס הראשון והשני מקובצים אל השלישי ביחס הראשון והשלישי מקובצים אל השני הוא יותר מאחד שלם] | ||||||||||||||||||||||||||||||||||||||||||||||||
|
שאלה יחס השני אל הנשאר מהשלישי כשחוסר ממנו הראשון מספר מונח ויחס השלישי אל הנשאר מהשני כשחוסר ממנו הראשון מספר אחר מונח | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ראוי שתוציא שלשה מספרים ינהגו כמו המנהג הקודם זה באלו היחסים הנזכרים הנה הוא | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והמספר הראשון הוא יהיה הראשון הנה והראשון והשני מקובצים יהיו המספר השני הנה | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
והמשל שיהיה יחס [880]השני אל הנשאר מהשלישי כשחוסר ממנו הראשון ג' שלמים ושליש ויחס השלישי אל הנשאר מהשני כשחוסר ממנו הראשון מספר ו' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה תוציא שלשה מספרים על הדרך הקודמת באלו היחסים הנזכרים הנה ר"ל שיהיה יחס מקובץ הראשון והשני אל השלישי ג' שלמים ושליש | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה הראשון [י"]ט | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והשני ד' ושליש | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והשלישי ז' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ולזה יהיה הראשון י"ט | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ותחבר עם השני י"ט ויהיו כ"ג שלמים ושליש והוא המספר השני | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ותחבר עם השלישי י"ט יהיו כ"ו והוא המספר השלישי | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והקש על זה | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וסבת זה מבוארת ממה שקדם | ||||||||||||||||||||||||||||||||||||||||||||||||
| You can also extract everything you extract by the previous method, i.e. if you know one of the numbers, you can find the other numbers after you extract the proportional numbers corresponding to the unknown numbers that meet these conditions and any consequent knowledge that enables to extract them. | ומזה גם כן תוכל להוציא כל מה שהוצאת בדרך הקודמת ר"ל שאם נודע לך אחד מהמספרים תוכל לדעת האחרים אחר שהוצאת המספרים שינהגו זה המנהג אשר הם גיליים למספרים הנעלמים ומה שנמשך לזה מהוצאתם מצד הידיעות אשר אפשר לקנות מהם זאת הידיעה | ||||||||||||||||||||||||||||||||||||||||||||||||
|
שאלה חברנו הראשון עם השני והיה יחס המקובץ אל הנשאר מהשלישי כשחוסר ממנו הראשון מספר מונח וכאשר חברנו הראשון עם השלישי היה יחס המקובץ אל הנשאר מהשני כשחוסר ממנו הראשון מספר אחר מונח | ||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה ראוי שתוציא לפי אלו היחסים שלשה מספרים על הדרך הקודמת | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וחצי המספר הראשון הוא יהיה המספר הראשון הנה והשני מחובר עם הראשון הזה רצוני חצי הראשון הקודם הוא יהיה המספר השני הנה | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
והמשל שיהיה יחס הראשון והשני מקובצים אל הנשאר [מהשלישי כשחוסר ממנו הראשון ד' שלמים וחצי ויחס הראשון והשלישי מקובצים אל הנשאר] מהשני כשחוסר ממנו הראשון הוא ה' שלמים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה נוציא המספרים השלשה על הדרך הקודמת לפי אלו היחסים רצוני שיהיה יחס הראשון והשני מקובצים אל השלישי ד' וחצי ויהיה יחס הראשון והשלישי מקובצים אל השני מספר ה' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
ולזה יהיה הראשון [881]כ"א וחצי | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והשני ה' וחצי | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והשלישי ו' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה חצי הראשון הוא י' וג' רביעיות והוא הראשון | ||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה חברנו י' וג' רביעיות עם השני ועלה י"ו ורביע והוא השני | ||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה חברנו י' וג' רביעיות עם השלישי ועלה י"ו וג' רביעיות והוא השלישי הנה | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והקש על זה | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וסבת זה מבוארת ממה שקדם | ||||||||||||||||||||||||||||||||||||||||||||||||
| Once you know the numbers that meet this condition, you can extract from them their proportional numbers based on the previous procedure. | ואחר שידעת המספרים שינהגו זה המנהג תוכל לקנות מהם הידיעה במספרים הגיליים על הצד הקודם | ||||||||||||||||||||||||||||||||||||||||||||||||
| Meaning, given the information about one of the proportional numbers, or the sum of two of them, or other kinds of information mentioned before, from which we can derive the knowledge of the proportional numbers corresponding to the three given numbers. | רצוני עם קדימת הידיעה באחד מהם ידוע הגיליות או מקובץ שנים מהם או שאר מיני הידיעות הנזכרות קודם אשר נעמד מהם על ידיעת המספרים הגיליים לשלשה המספרים המונחים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
שאלה המספר הראשון עם חלק מונח מהשני והשלישי מקובצים שוה אל המספר השני עם חלק אחד מונח מהראשון והשלישי מקובצים וכן הוא שוה אל המספר השלישי עם חלק אחר מונח מהראשון והשני מקובצים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה ראוי שתוציא תחלה המספרים שינהגו זה המנהג על הצד שהתבאר במאמר הראשון מזה הספר | ||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
וממה שקדם לך מהידיעה באחד מהמספרים הגיליים תעמוד על ידיעת המספרים הגיליים בכללם איש על מקומו והמספרים ההם הם המבוקשים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והמשל שיהיה הראשון עם רביעית הנשארים שוה אל השני עם ששית הנשארים והוא גם כן שוה אל השלישי עם תשיעית הנשארים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וכבר ידענו שהמספרים אשר ינהגו כמו זה המנהג הראשון מהם קי"ט והשני קנ"א והשלישי קס"ט | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
[והמספר] [882]שהוא הגיליי לשני הוא כ' | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ו[נוציא] שאר הגיליים על זה היחס | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ולזה יהיה הגיליי לראשון ט"ו שלמים וקט"ו חלקים מקנ"א באחד שלם והוא הראשון הנה | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ויהיה הגיליי לשלישי כ"ב שלמים ונ"ח חלקים מקנ"א באחד והוא השלישי הנה | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה המספרים המבוקשים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תרצה תוכל לבחון זה | ||||||||||||||||||||||||||||||||||||||||||||||||
|
והיה זה כן לפי שהנמשכים על יחס הקודמים הנה יהיה יחס הראשון מהקודמים אל השני מהם כיחס הראשון מהנמשכים אל השני מהם | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ולזה יהיה יחס הראשון מהקודמים אל השלישי כיחס הראשון מהנמשכים אל השלישי | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר קבצנו הנה יהיה יחס הראשון מהקודמים אל השני והשלישי מהם מקובצים כמו יחס הראשון מהנמשכים אל השני והשלישי מהם מקובצים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ולזה יהיה יחס הראשון מהקודמים אל רביעית השני והשלישי מהם [מקובצים כמו יחס הראשון מהנמשכים אל רביעית השני והשלישי מהם] | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר קבצנו הנה יהיה יחס הראשון מהקודמים עם רביעית השני והשלישי מהם אל הראשון מהקודמים כיחס הראשון מהנמשכים עם רביעית השני והשלישי אל הראשון מהנמשכים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ובזה התבאר שיחס השני מהקודמים עם ששית השלישי והראשון מהם אל השני מהקודמים הוא כמו יחס השני מהנמשכים עם ששית השלישי והראשון מהם אל השני מהנמשכים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ובזה התבאר שיחס השלישי מהקודמים עם תשיעית הנשארים מהם[883] אל השלישי מהקודמים הוא כמו יחס השלישי מהנמשכים עם תשיעית הנשארים מהם אל השלישי מהנמשכים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר המירונו הנה יחס הראשון מהקודמים עם הרביעית הנשארים מהם אל הראשון מהנמשכים עם רביעית הנשארים מהם הוא כיחס הראשון מהקודמים אל הראשון מהנמשכים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ויחס השני מהקודמים עם ששית הנשארים מהם אל השני מהנמשכים עם ששית הנשארים מהם הוא כיחס השני מהקודמים אל השני מהנמשכים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
ויחס השלישי מהקודמים עם תשיעית הנשארים מהם אל השלישי מהנמשכים עם תשיעית הנשארים מהם הוא כיחס השלישי מהקודמים אל השלישי מהנמשכים | ||||||||||||||||||||||||||||||||||||||||||||||||
|
[884][ואולם יחס הראשון מהקודמים אל] הראשון מהנמשכים הוא [כיחס השני אל השני וכיחס השלישי אל] השלישי | ||||||||||||||||||||||||||||||||||||||||||||||||
|
אם כן יחס הראשון [מהקודמים עם רביעית הנשארים מהם אל הראשון מהנמשכים עם רביעית] הנשארים מהם הוא כמו יחס השני מהקודמי[ם עם ששית הנשארים מהם אל השני מהנמשכים עם ששית הנשארים מהם וכמו יחס השלישי מהקודמים עם תשיע]ית הנשארים מהם אל השלישי מהנמשכים עם תשיעית הנשארים מהם | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר המירונו יהיו גם כן מתיחסים אבל הקודמים כלם שוים הנה [הנמשכים] כלם שוים והקש על זה | ||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||
| By this you can extract the proportional numbers corresponding to the three known numbers, using any knowledge concerning these unknown numbers, meaning, if you know the excess of one unknown over another unknown, or if you know the sum of two of the unknowns, and any consequent kind of knowledge that enables to find the unknown numbers according to the method explained above. | ובזה תוכל להוציא מאי זו ידיעה שתהיה לך באלו המספרים הנעלמים המספרים הגיליים למספרים השלשה הידועים רצוני אם ידעת יתרון אחד מהנעלמים ידועי הגיליות [על אחד מהם ידוע הגיליות] או אם ידעת מספר שנים מהנעלמים מקובצים ומה שימשך לו מאופני [הידיעה] יהיה אפשר לקנות ממנה המספרים הנעלמים על הצד שהתבאר קודם | ||||||||||||||||||||||||||||||||||||||||||||||||
| Understand and you will find. | ותבין ותמצא | ||||||||||||||||||||||||||||||||||||||||||||||||
Colophon |
|||||||||||||||||||||||||||||||||||||||||||||||||
| Edition I | |||||||||||||||||||||||||||||||||||||||||||||||||
|
כתב המחבר נשלם השער הששי מזה המאמר ובהשלמו נשלם זה הספר | ||||||||||||||||||||||||||||||||||||||||||||||||
| והתהלה לאל לבדו | |||||||||||||||||||||||||||||||||||||||||||||||||
|
והיתה השלמתו בראש ניסן שנת שמנים ואחת לפרט האלף הששי | ||||||||||||||||||||||||||||||||||||||||||||||||
|
בהגיעי לשנת שלשים ושלש משנותי | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וברוך העוזר | ||||||||||||||||||||||||||||||||||||||||||||||||
| Edition II | |||||||||||||||||||||||||||||||||||||||||||||||||
|
נשלם השער השישי מזה המאמר ובהשלמו נשלם זה הספר | ||||||||||||||||||||||||||||||||||||||||||||||||
| והתהלה לאל לבדו | |||||||||||||||||||||||||||||||||||||||||||||||||
|
והיתה השלמתו בחודש אלול של שנת שמונים ושתים לפרט האלף השישי | ||||||||||||||||||||||||||||||||||||||||||||||||
|
וברוך העוזר | ||||||||||||||||||||||||||||||||||||||||||||||||
Notes
Appendix I: Glossary of Terms
| rank | מדרגה, מעלה |
Appendix II: Bibliography
Levi Ben Gershon (called also: Leo Hebraus, Leo de Balneolis, Maestro Leon; known today as Gersonides)
b. 1288, Bagnols, Provence – d. 1344, Provence
Sefer Ma‛ase Hoshev
1321-2
Manuscripts:
- 1) Jerusalem, The National Library of Israel Ms. Heb. 8°2005 (IMHM: B 400 (8°2005)), (1410)
- [J2005]
- 2) London, British Library Or. 10547 (IMHM: f 7909), ff. 1-118 (14th-15th century)
- [L10547]
- 3) Moscow, Russian State Library, Ms. Guenzburg 30/3 (IMHM: f 6711), ff. 40r-122v (1503)
- [Mo30]
- 4) Moscow, Russian State Library, Ms. Guenzburg 1063 (IMHM: f 48133), ff. 1-84 (15th century)
- 5) München, Bayerische Staatsbibliothek, Cod. hebr. 36/1 (IMHM: f 1166), ff. 1-9 (1485)
- [Mu36]
- 6) München, Bayerische Staatsbibliothek, Cod. hebr. 68/6 (IMHM: f 1131), ff. 376r-432r (Roma, 1552)
- [Mu68]
- 7) New York, Jewish Theological Seminary Ms. 2624/2 (IMHM: f 28877), ff. 11r-59v (16th century)
- [N2624]
- 8) Paris, Bibliothèque Nationale de France heb. 1029/6 (IMHM: f 15721), ff. 236r-295v (15th-16th century)
- 9) Parma, Biblioteca Palatina Parm. 2271 (IMHM: f 13179), (14th century)
- [Parm2271]
- 10) Parma, Biblioteca Palatina Parm. 2462 (IMHM: f 13466), (14th-15th century)
- [Parm2462]
- 11) Vatican, Biblioteca Apostolica ebr. 399/1 (IMHM: f 477), ff. 1r-23v (15th century)
- [V399]
The transcript is based mainly on manuscript Parma 2271
Edition:
- Levi ben Gershon. Sefer Maassei Chosheb: Die Praxis des Rechners, Ein hebräisch-arithmetisches Werk des Levi ben Gerschom aus dem Jahre 1321. Ed. Gerson Lange. Frankfurt am Main: Louis Golde, 1909.
Bibliography:
- Carlebach, Joseph. 1910. Lewi ben Gerson als Mathematiker: ein Beitrag zur Geschichte der Mathematik bei den Juden. Berlin: L. Lamm.
- Chemla, Karine and Serge Pahaut. 1992. Remarques sur les ouvrages mathématiques de Gersonide. In: Freudenthal 1992, pp. 149–191.
- Freudenthal, Gad ed. 1992. Studies on Gersonides: A Fourteenth-Century Jewish Philosopher-Scientist. Leiden: Brill.
- Kellner, Menachem. 1992. An Annotated List of Writings by and about R. Levi ben Gershom, in Freudenthal 1992, pp. 367-414.
- Langermann, Tzvi and Shai Simonson. 2000. The Hebrew Mathematical Tradition. In: Helaine Seline ed. Mathematics Across Cultures. Dordrecht: Kluwer, pp. 167–188.
- Rabinovitch, Nahum. 1970. Rabbi Levi ben Gershon and the Origins of Mathematical Induction, Archive for History of Exact Sciences 6 , pp. 237-248.
- Rashed, Roshdi. 1994. The Development of Arabic Mathematics: Between Arithmetic and Algebra. Translated by A.F.W. Armstrong. Dordrecht, Boston, London: Kluwer Academic Publishers, pp. 65-84.
- Sarfatti, Gad ben ‛Ami. 1968. Mathematical Terminology in Hebrew Scientific Literature of the Middle Ages. Jerusalem: Magnes Press, pp. 220-227.
- Simonson, Shai. 2000a. The Missing Problems of Gersonides. A Critical Edition, Historia Mathematica 27 (3), pp. 243–302 and 27 (4), pp. 384–431.
- ———.2000b. Mathematical Gems of Levi ben Gershon, Mathematics Teacher 93, pp. 659-663.
- ———. 2000c. The Mathematics of Levi ben Gershon, the Ralbag, BDD (Bekhol Derakhekha Daehu= בכל דרכיך דעהו) 10 (2000), pp.5-21.
- Steinschneider, Moritz. 1893-1901. Mathematik bei den Juden. Berlin-Leipzig-Frankfurt: Kaufmann, pp. 129-133 (e103-e107); repr. Hildesheim: G. Olms, 1964 and 2001.
- Weil-Guény, Anne-Marie. 1992. Gersonide en son temps: un tableau chronologique. In: Freudenthal 1992, pp. 355–365.
- Yadegari, Mohammad. 1978. The Use of Mathematical Induction by Abū Kāmil Shujā‛ Ibn Aslam (850-930), Isis 69, 2 (Jun. 1978), pp. 259-262.
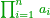
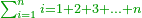
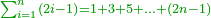
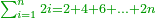
![\scriptstyle{\color{OliveGreen}{\sum_{i=1}^n \sum_{j=i}^n=\left(1+2+3+\ldots+n\right)+\left(2+3+\ldots+n\right)+\ldots+\left[\left(n-1\right)+n\right]+n}}](/mediawiki/images/math/5/a/7/5a78172676e910bb15617d2cf0bd669c.png)
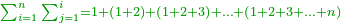


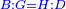

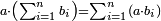

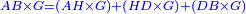
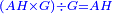
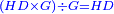
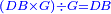
![\scriptstyle{\color{blue}{\left[\left(AH\times G\right)+\left(HD\times G\right)+\left(DB\times G\right)\right]\div G=AB}}](/mediawiki/images/math/8/e/4/8e4326a58d9683b670dac49dc960d905.png)
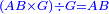

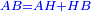

![\scriptstyle{\color{blue}{\left[AH\times\left(GZ+ZC+CD\right)\right]+\left[HB\times\left(GZ+ZC+CD\right)\right]=AB\times GD}}](/mediawiki/images/math/4/4/3/443b9e3c2cc8c4491100b2c17f3d419b.png)
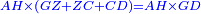
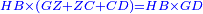
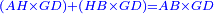
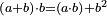
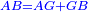
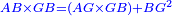
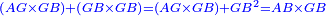
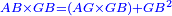
![\scriptstyle\left[\left(a+b\right)\sdot b\right]+\left(\frac{1}{2}\sdot a\right)^2=\left[\left(\frac{1}{2}\sdot a\right)+b\right]^2](/mediawiki/images/math/a/0/b/a0b403dd8b7c15a5cb1dc47a5f8ca5bb.png)
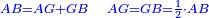
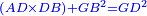
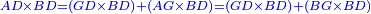
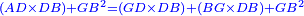
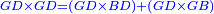
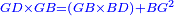
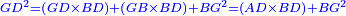
![\scriptstyle\left(a+b\right)^2=a^2+b^2+\left[2\sdot\left(a\sdot b\right)\right]](/mediawiki/images/math/7/2/7/727b0c35fd93a7a347bed8413905049f.png)
![\scriptstyle{\color{blue}{AG^2=AB^2+BG^2+\left[2\sdot\left(AB\times BG\right)\right]}}](/mediawiki/images/math/b/f/2/bf236f40372404bbffe0b590649560ce.png)



![\scriptstyle\left(a+b\right)^2=\left[a\sdot\left(a+b\right)\right]+\left(a\sdot b\right)+b^2](/mediawiki/images/math/6/d/4/6d450eeb47934cad7f1a81a10ccacaf6.png)
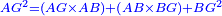
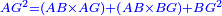
![\scriptstyle\left[\frac{1}{2}\sdot\left(a+b\right)\right]^2=\left(a\sdot b\right)+\left[b-\left[\frac{1}{2}\sdot\left(a+b\right)\right]\right]^2=\left(a\sdot b\right)+\left[\left[\frac{1}{2}\sdot\left(a+b\right)\right]-a\right]^2](/mediawiki/images/math/3/1/c/31c3d3d1db8bc18e36c5dee2c30742ba.png)
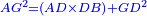
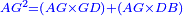
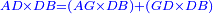
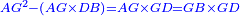
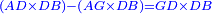
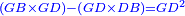
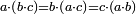

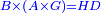
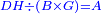

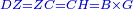
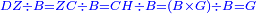
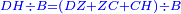


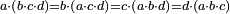
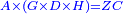
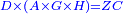


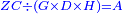

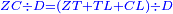

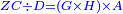

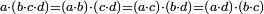



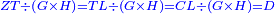
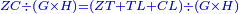
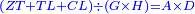
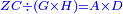
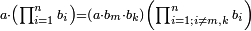
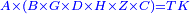

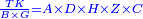
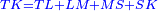

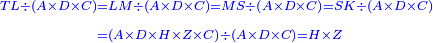
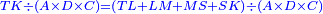
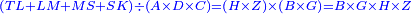
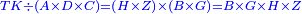
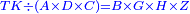






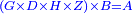
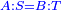



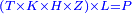

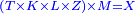
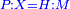
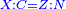
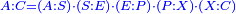





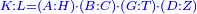
 is relatively prime to
is relatively prime to 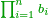 , then
, then  for each i=1,2,…,n]
for each i=1,2,…,n]
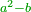 is relatively prime to all integers less than
is relatively prime to all integers less than  .
.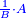 ]; H parts of G [
]; H parts of G [ ] of the remainder; and Z parts of D [
] of the remainder; and Z parts of D [ ] of its remainder.
] of its remainder. ] with one part of B [
] with one part of B [ ] of the remainder and H parts of G [
] of the remainder and H parts of G [
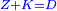
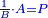
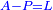
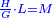

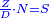

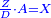
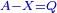

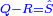
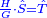
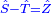
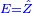
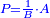
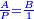
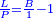
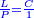


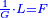
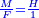


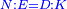


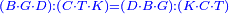
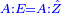
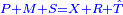
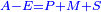
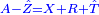
![\scriptstyle{\color{blue}{\left[\frac{H}{G}\sdot\left(A\times B\right)\right]\sdot\frac{Z}{D}}}](/mediawiki/images/math/c/8/2/c82c2729bf4a1918b532560a86354d0a.png)
![\scriptstyle{\color{blue}{\left[\left(\frac{Z}{D}\sdot A\right)\sdot B\right]\sdot\frac{H}{G}=\left[\frac{H}{G}\sdot\left(A\times B\right)\right]\sdot\frac{Z}{D}}}](/mediawiki/images/math/8/9/5/89564b2593293f096bdcde0cfd8cef3f.png)
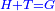

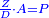








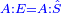
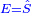
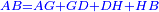


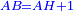
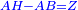
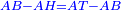


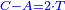
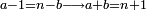


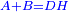

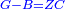

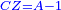
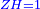
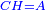
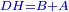
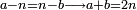

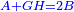



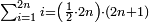

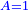

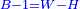

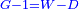
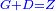
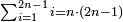
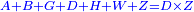
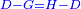
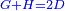
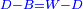
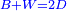

![\scriptstyle\left[\frac{1}{2}\sdot\left(2n-1\right)\right]\sdot2n=\sum_{i=1}^{2n-1} i](/mediawiki/images/math/b/b/3/bb32b7826dec5f835576e6e2ce3e99ea.png)
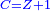
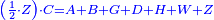
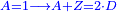
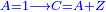

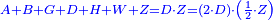

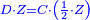
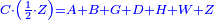
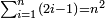
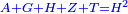
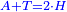

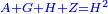
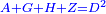

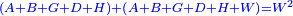
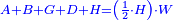
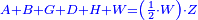
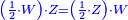
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle\left(A+B+G+D+H\right)+\left(A+B+G+D+H+W\right)&\scriptstyle=\left[\left(\frac{1}{2}\sdot H\right)\sdot W\right]+\left[\left(\frac{1}{2}\sdot Z\right)\sdot W\right]\\&\scriptstyle=\left[\frac{1}{2}\sdot\left(H+Z\right)\right]\sdot W\\\end{align}}}](/mediawiki/images/math/c/4/e/c4e931e2a1c2f16a524da4164f945682.png)
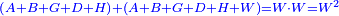
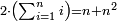
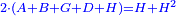


![\scriptstyle{\color{OliveGreen}{\sum_{i=1}^{n} i=\left[\frac{1}{2}\sdot\left(n^2\right)\right]+\left(\frac{1}{2}\sdot n\right)}}](/mediawiki/images/math/5/d/d/5dd9bdcf55fc0efe023ed904988894b8.png)

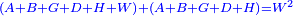
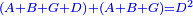

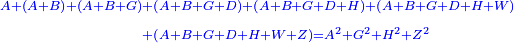

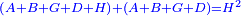
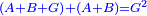

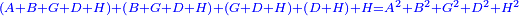
![\scriptstyle{\color{blue}{\begin{align}&\scriptstyle\left[\left(A+B+G+D+H\right)+\left(B+G+D+H\right)+\left(G+D+H\right)+\left(D+H\right)+H\right]\\&\scriptstyle+\left[A+\left(A+B\right)+\left(A+B+G\right)+\left(A+B+G+D\right)\right]=H\sdot\left(A+B+G+D+H\right)\\\end{align}}}](/mediawiki/images/math/a/6/2/a62b2b4b991bc160e31322d6f0ab7b69.png)
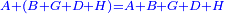
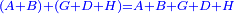
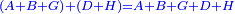
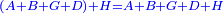
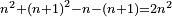


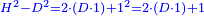

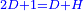

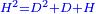
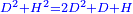
![\scriptstyle{\color{blue}{\left[\left(A+B+G+D+H\right)+\left(B+G+D+H\right)+\left(G+D+H\right)+\left(D+H\right)+H\right]-\left(A+B+G+D+H\right)}}](/mediawiki/images/math/b/1/c/b1c5db7125cf0910183a167992d5b4ac.png)
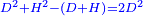
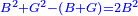
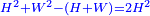
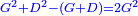
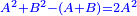
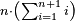
![\scriptstyle{\color{blue}{\begin{align}&\scriptstyle W\times\left(A+B+G+D+H+W\right)\\&\scriptstyle=\left[A+\left(A+B\right)+\left(A+B+G\right)+\left(A+B+G+D\right)+\left(A+B+G+D+H\right)\right]\\&\scriptstyle+\left(A+B+G+D+H+W\right)+\left(B+G+D+H+W\right)+\left(G+D+H+W\right)\\&\scriptstyle+\left(D+H+W\right)+\left(H+W\right)+W\\\end{align}}}](/mediawiki/images/math/a/3/b/a3ba61814919c6cc05cd637ee19155ef.png)
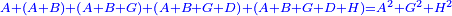

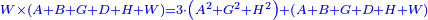
![\scriptstyle\left[n-\left[\frac{1}{3}\sdot\left(n-1\right)\right]\right]\sdot\left(\sum_{i=1}^{n} i\right)=\sum_{i=1}^{n} i^2](/mediawiki/images/math/f/2/d/f2d374bf634bb323ade34d5e3b40f80c.png)
![\scriptstyle{\color{blue}{\begin{align}&\scriptstyle W\times\left(A+B+G+D+H+W\right)\\&\scriptstyle=\left(A+B+G+D+H+W\right)+\left(B+G+D+H+W\right)+\left(G+D+H+W\right)\\&\scriptstyle+\left(D+H+W\right)+\left(H+W\right)+W\\&\scriptstyle+\left[A+\left(A+B\right)+\left(A+B+G\right)+\left(A+B+G+D\right)+\left(A+B+G+D+H\right)\right]\\\end{align}}}](/mediawiki/images/math/0/0/5/005c49f1f729dd8537fb46774cee24e5.png)
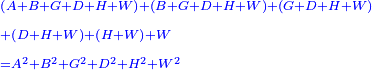
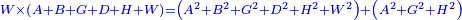
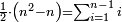
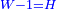


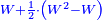
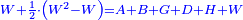
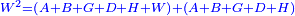
![\scriptstyle{\color{blue}{\begin{align}&\scriptstyle W+\frac{1}{2}\sdot\left[\left[\left(A+B+G+D+H+W\right)+\left(A+B+G+D+H\right)\right]-W\right]\\&\scriptstyle=W+\left(A+B+G+D+H\right)\\&\scriptstyle=A+B+G+D+H+W\\\end{align}}}](/mediawiki/images/math/f/3/b/f3bff66633d181a09dcb6fd6527dc6c4.png)



![\scriptstyle{\color{blue}{H\sdot\left[\left(A+B+G+D\right)+\left(A+B+G+D+H\right)\right]=H^3}}](/mediawiki/images/math/a/1/f/a1f60d9f554082c02d745e3faac3dc7a.png)
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle H\sdot\left[\left(A+B+G+D\right)+\left(A+B+G+D+H\right)\right]&\scriptstyle=\left(H\sdot H\right)+H\sdot\left[\left(A+B+G+D\right)+\left(A+B+G+D\right)\right]\\&\scriptstyle=H^2+\left[2\sdot H\sdot\left(A+B+G+D\right)\right]\\\end{align}}}](/mediawiki/images/math/7/5/f/75f0f2ac673371a6e1a9870f6a9b5724.png)
![\scriptstyle{\color{blue}{H^3=H^2+\left[2\sdot H\sdot\left(A+B+G+D\right)\right]}}](/mediawiki/images/math/3/0/f/30f23782fa5f2bc3bdf71b0a49bc5b58.png)
![\scriptstyle{\color{blue}{\left(A+B+G+D+H\right)^2=H^2+\left[2\sdot H\sdot\left(A+B+G+D\right)\right]+\left(A+B+G+D\right)^2}}](/mediawiki/images/math/c/7/1/c71b31d3d9b03f2498917df7556a32c2.png)
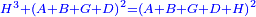
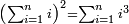
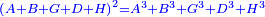

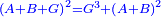
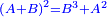
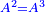

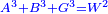

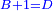

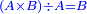
![\scriptstyle{\color{blue}{\left[\left(A\times B\right)+A\right]\div A=B+1=D}}](/mediawiki/images/math/4/c/7/4c72e6086f27ad82de33c0f13d91b7de.png)
![\scriptstyle a<b<c\longrightarrow\left[\left[c\sdot\left(b-a\right)\right]+\left[a\sdot\left(c-b\right)\right]\right]\div b=c-a](/mediawiki/images/math/5/d/e/5dec26d71defb15bec252b4453befdb1.png)


![\scriptstyle{\color{blue}{\left[\left(G\times D\right)+\left(A\times H\right)\right]\div B=H+D=G-A}}](/mediawiki/images/math/6/4/a/64ae4b5f02d621d9b7803f7aa03518d1.png)
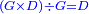
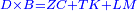
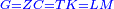


![\scriptstyle 2\sdot\left[\left(a-2\right)\sdot\left(b-1\right)\right]+b+\left(a-2\right)+\left(b-a\right)=2\sdot\left[\left(a-1\right)\sdot\left(b-1\right)\right]](/mediawiki/images/math/1/8/4/18450d37c563f2e06d0261297caca2a6.png)
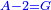
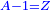



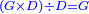
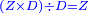


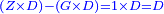
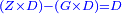
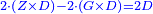
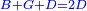
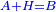
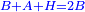
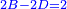

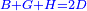
![\scriptstyle\left(b-a\right)+\left(a\sdot b\right)=\left(b-1\right)+b+\left[\left(a-1\right)\sdot\left(b-1\right)\right]](/mediawiki/images/math/f/b/a/fba98b9b0fec6515a8c1a04c97ba7587.png)
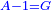
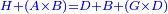
![\scriptstyle{\color{blue}{\left[\left(G\times D\right)+D\right]\div A=D}}](/mediawiki/images/math/3/c/b/3cb157a576f2cf89e7f328c4607ff2c8.png)

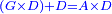

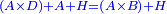
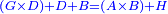
![\scriptstyle 2\sdot\left[\left(b-1\right)\sdot\left(a-2\right)\right]+b+\left(a-2\right)+\left(b-a\right)=\left[\left(a-1\right)\sdot b\right]+\left[\left(b-1\right)\sdot\left(a-2\right)\right]+\left(b-a\right)](/mediawiki/images/math/0/4/b/04b8bdb4c5777310cbe43bd2eb806ca4.png)
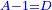
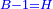
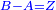
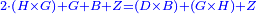
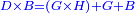
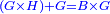

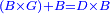
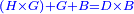
![\scriptstyle2\sdot\left[\left(a-1\right)\sdot\left(b-c\right)\right]+\left[a\sdot\left(c-2\right)\right]+\left[\left(a-1\right)\sdot\left(c-2\right)\right]+a+\left(b-c\right)+\left(a-b\right)=2\sdot\left[\left(b-1\right)\sdot\left(a-1\right)\right]](/mediawiki/images/math/d/2/0/d20cf3cbc9a55e98a8a825c85dd8837c.png)


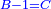

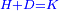
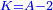
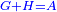

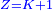

![\scriptstyle{\color{blue}{\left[2\sdot\left(C\times H\right)+\left(D\times B\right)+\left(D\times C\right)\right]+B+H+T=2\sdot\left(Z\times C\right)}}](/mediawiki/images/math/c/9/8/c9861a74c492a80903358f156464c331.png)
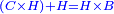
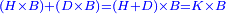
![\scriptstyle{\color{blue}{\left(H\times B\right)+\left(D\times B\right)+B=\left[\left(H+D\right)\times B\right]+B=\left(K\times B\right)+B=Z\times B}}](/mediawiki/images/math/8/f/3/8f3056def97c18c383e8672c0fbdbcc8.png)
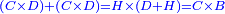
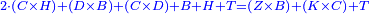
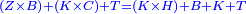
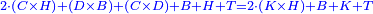
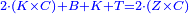

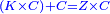
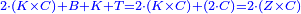
![\scriptstyle{\color{blue}{\left[2\sdot\left(H\times C\right)+\left(D\times B\right)+\left(C\times D\right)\right]+B+H+T=2\sdot\left(Z\times C\right)}}](/mediawiki/images/math/d/d/5/dd518b4ffa5a2c341a3e6fe9c1b2f851.png)
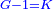
![\scriptstyle{\color{blue}{\left[\left[2\sdot\left(C\times H\right)\right]+\left[D\times\left(A\times B\right)\right]+\left(T\times B\right)+B+H\right]\div\left(Z\times C\right)=G}}](/mediawiki/images/math/4/d/2/4d2307620b80b7e31106780c8cd37027.png)

![\scriptstyle{\color{blue}{\left[D\times\left(A\times B\right)\right]+\left(D\times T\right)=\left[D\times\left(Z\times H\right)\right]+\left(D\times B\right)+\left(D\times C\right)}}](/mediawiki/images/math/d/0/c/d0ce15cbd96bca73c3bacf072c5c030b.png)
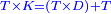
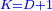
![\scriptstyle{\color{blue}{\left[D\times\left(A\times B\right)\right]+\left(T\times B\right)=\left[D\times\left(Z\times C\right)\right]+\left(D\times K\right)+\left(D\times C\right)+T}}](/mediawiki/images/math/5/8/a/58ab42efb9a1ebd19307e337fff5b8c9.png)
![\scriptstyle{\color{blue}{\left[2\sdot\left(C\times H\right)\right]+\left(D\times K\right)+\left(D\times C\right)+B+H+T=Z\times C}}](/mediawiki/images/math/1/f/a/1fa4172b8c4a8d5b6444caf941e78203.png)
![\scriptstyle{\color{blue}{\left[\left[2\sdot\left(C\times H\right)\right]+\left[D\times\left(A\times B\right)\right]+\left(T\times B\right)+B+H\right]\div\left(Z\times C\right)=D+2}}](/mediawiki/images/math/0/8/c/08cb2f16b4aa493ad2fc89d05c9897b8.png)

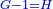
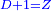
![\scriptstyle{\color{blue}{\left[\left(D\times H\right)+G+D\right]\div G=Z}}](/mediawiki/images/math/e/a/a/eaa123c9bf2888c01380231a126fb1ce.png)
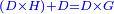
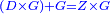
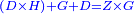

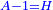
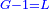
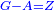
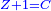
![\scriptstyle{\color{blue}{\left[\left(D\times L\right)+\left(T\times H\right)+G+D\right]\div B=C}}](/mediawiki/images/math/e/4/9/e494530244b46f899acc4c6e32ea0668.png)
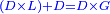


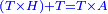

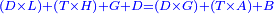
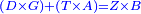
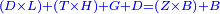
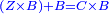
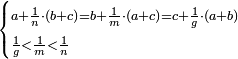
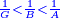




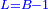


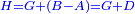
![\scriptstyle{\color{blue}{T=H+2\sdot\left[\left(G-1\right)\sdot\left(B-A\right)\right]=H+2\sdot\left(D\sdot C\right)}}](/mediawiki/images/math/b/f/b/bfb9c4010cb4a0e219e48655de275732.png)
![\scriptstyle{\color{blue}{K=T+2\sdot\left[\left(A-1\right)\sdot\left(G-B\right)\right]=T+2\sdot\left[Z\sdot\left(A-1\right)\right]}}](/mediawiki/images/math/c/f/d/cfdc1f166ed70f148002f168c6e0b901.png)
![\scriptstyle{\color{OliveGreen}{\begin{cases}\scriptstyle a=g+\left(m-n\right)\\\scriptstyle b=a+\left[2\sdot\left(g-1\right)\sdot\left(m-n\right)\right]\\\scriptstyle c=b+\left[2\sdot\left(n-1\right)\sdot\left(g-m\right)\right]\end{cases}}}](/mediawiki/images/math/6/6/d/66d080548de2822e9d6c4b521f157f79.png)

![\scriptstyle{\color{blue}{K=H+2\sdot\left(D\sdot C\right)+2\sdot\left[Z\sdot\left(A-1\right)\right]=H+2\sdot\left(D\sdot C\right)+\left(2\sdot Z\right)}}](/mediawiki/images/math/1/b/a/1babc19de40333240181caf13d2fa2bd.png)
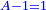
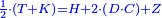

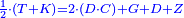
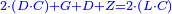
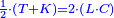
![\scriptstyle{\color{blue}{T+K=2\sdot\left[2\sdot\left(L\sdot C\right)\right]}}](/mediawiki/images/math/4/e/8/4e8d79609286a4f5bad858bad6b60d38.png)
![\scriptstyle{\color{blue}{T+K=A\sdot\left[2\sdot\left(L\sdot C\right)\right]}}](/mediawiki/images/math/a/0/7/a074f250d619c16dc64f8747ee376da3.png)


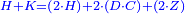
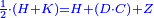
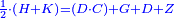
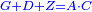
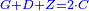
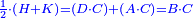

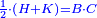

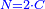
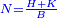
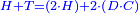
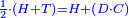
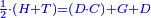

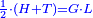


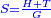

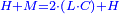
![\scriptstyle{\color{blue}{T+N=H+\left[2\sdot\left(D\sdot C\right)\right]+\left(2\sdot C\right)}}](/mediawiki/images/math/1/6/4/1640af4c164313c3d187ed941cb25bf7.png)

![\scriptstyle{\color{blue}{\left[2\sdot\left(D\sdot C\right)\right]+\left(2\sdot C\right)=2\sdot\left(L\sdot C\right)}}](/mediawiki/images/math/4/1/9/419778f5f2d4e82a4db9bf69f899c59e.png)

![\scriptstyle{\color{blue}{K+S=H+\left[2\sdot\left(D\sdot C\right)\right]+{\color{red}{\left(2\sdot Z\right)}}+\left(2\sdot L\right)}}](/mediawiki/images/math/9/a/0/9a08ef126d84a5ac77fa6688b26f218e.png)

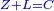
![\scriptstyle{\color{blue}{K+S=H+\left[2\sdot\left(D\sdot C\right)\right]+\left[2\sdot\left(Z+L\right)\right]=H+\left[2\sdot\left(D\sdot C\right)\right]+\left(2\sdot C\right)}}](/mediawiki/images/math/4/d/a/4da002ab6b4816c22134163fcafcfdc5.png)
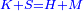






![\scriptstyle{\color{blue}{M=\left[\left(B\sdot G\right)\sdot\left(A-2\right)\right]+G+\left(B-A\right)=\left[\left(B\sdot G\right)\sdot D\right]+G+H}}](/mediawiki/images/math/a/2/7/a277055131b240101d2d6e6d30e28a9d.png)
![\scriptstyle{\color{blue}{N=M+\left[2\sdot\left[\left(G-1\right)\sdot\left(B-A\right)\right]\right]=M+2\sdot\left(H\sdot L\right)}}](/mediawiki/images/math/2/3/e/23ed406d1d7ede8b7085612676f97b0e.png)
![\scriptstyle{\color{blue}{S=N+\left[2\sdot\left[\left(A-1\right)\sdot\left(G-B\right)\right]\right]=N+2\sdot\left(Z\sdot C\right)}}](/mediawiki/images/math/e/9/d/e9daa6b44f26218b7dd5e1452c419861.png)
![\scriptstyle{\color{OliveGreen}{\begin{cases}\scriptstyle a=g+\left(m-n\right)+\left[\left(n-2\right)\sdot m\sdot g\right]\\\scriptstyle b=a+\left[2\sdot\left(g-1\right)\sdot\left(m-n\right)\right]\\\scriptstyle c=b+\left[2\sdot\left(n-1\right)\sdot\left(g-m\right)\right]\end{cases}}}](/mediawiki/images/math/0/7/c/07c18907863e5edcaceddde1ee1d9cd3.png)
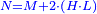

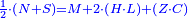
![\scriptstyle{\color{blue}{M=\left[D\sdot\left(B\sdot G\right)\right]+G+H}}](/mediawiki/images/math/8/7/a/87a2e3b71caf43dec06fe29a36790be0.png)
![\scriptstyle{\color{blue}{\frac{1}{2}\sdot\left(N+S\right)=\left[D\sdot\left(B\sdot G\right)\right]+2\sdot\left(H\sdot L\right)+\left(Z\sdot C\right)+G+H}}](/mediawiki/images/math/6/a/7/6a787e87628d74fe5cdd5d6be89ca64f.png)
![\scriptstyle{\color{blue}{\left[D\sdot\left(B\sdot G\right)\right]+2\sdot\left(H\sdot L\right)+\left(Z\sdot C\right)+G+H=A\sdot\left(T\sdot L\right)}}](/mediawiki/images/math/3/c/7/3c793b940ec0d2566de750092c46627c.png)
![\scriptstyle{\color{blue}{N+S=A\sdot\left[2\sdot\left(T\sdot L\right)\right]}}](/mediawiki/images/math/c/f/4/cf46eb0e8d71f42bfdae9f7f76d9fac4.png)
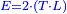
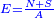
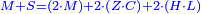
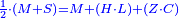
![\scriptstyle{\color{blue}{\frac{1}{2}\sdot\left(M+S\right)=\left(H\sdot L\right)+\left(Z\sdot C\right)+G+H+\left[D\sdot\left(B\sdot G\right)\right]}}](/mediawiki/images/math/1/3/9/1397754c6c98ee6fca5504c1093cb326.png)
![\scriptstyle{\color{blue}{\left(H\sdot L\right)+\left(Z\sdot C\right)+G+H=B\sdot\left[\left(G-A\right)+1\right]=B\sdot\left[\left(H+Z\right)+1\right]}}](/mediawiki/images/math/0/0/a/00af68ec467b23aa5beaed4aadde439e.png)
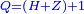
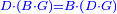
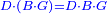
![\scriptstyle{\color{blue}{\frac{1}{2}\sdot\left(M+S\right)=B\sdot\left[\left(D\sdot G\right)+Q\right]}}](/mediawiki/images/math/2/0/8/2087ade4e08563e87fcd3fdcc31b340c.png)
![\scriptstyle{\color{blue}{M+S=B\sdot\left[2\sdot\left(D\sdot G\right)+\left(2\sdot Q\right)\right]}}](/mediawiki/images/math/e/8/e/e8e425c0814e097abbd8555db10d803b.png)

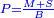
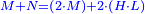
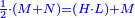
![\scriptstyle{\color{blue}{\frac{1}{2}\sdot\left(M+N\right)=\left(H\sdot L\right)+G+H+\left[D\sdot\left(B\sdot G\right)\right]}}](/mediawiki/images/math/8/1/b/81bed312d2aa4de43ae25feb2d6ebbbd.png)

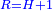
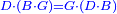
![\scriptstyle{\color{blue}{\frac{1}{2}\sdot\left(M+N\right)=G\sdot\left[\left(D\sdot B\right)+R\right]}}](/mediawiki/images/math/8/0/4/8042476e0b7b936abffee1933054397f.png)
![\scriptstyle{\color{blue}{M+N=G\sdot\left[2\sdot\left(D\sdot B\right)+\left(2\sdot R\right)\right]}}](/mediawiki/images/math/d/f/5/df53e76b109f98ca6124c40ea821c31f.png)
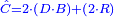
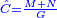
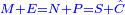
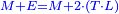

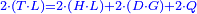

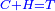
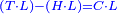


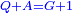

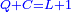

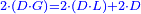

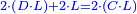
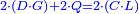
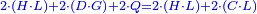
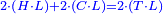

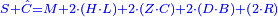
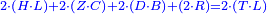
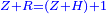
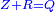
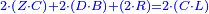

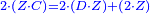
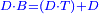
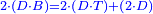
![\scriptstyle{\color{blue}{2\sdot\left(Z\sdot C\right)+2\sdot\left(D\sdot B\right)+\left(2\sdot R\right)=\left[2\sdot\left[D\sdot\left(Z+T\right)\right]\right]+2\sdot\left(Z+R+D\right)}}](/mediawiki/images/math/7/7/5/7757474cfc85eee10480be2bc5aba143.png)



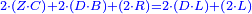
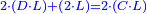
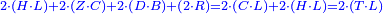
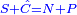
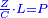
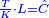
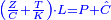
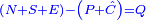
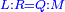
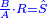
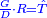

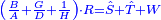
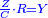

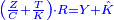

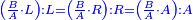
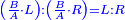
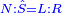
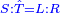
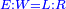
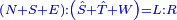
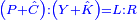

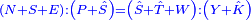
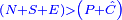
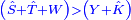

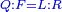
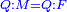
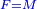
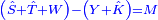
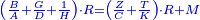
![\scriptstyle{\color{blue}{\left[B+\left(\frac{Z}{H}\sdot A\right)\right]-\left[A+\left[\left(\frac{G}{D}+\frac{1}{H}\right)\sdot B\right]\right]=T}}](/mediawiki/images/math/5/a/c/5ac859aabc89f643a2a32afc82ddf127.png)
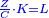
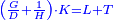
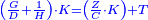
![\scriptstyle{\color{blue}{A+\left[\left(\frac{G}{D}+\frac{1}{H}\right)\sdot B\right]=M}}](/mediawiki/images/math/7/0/e/70e70a02417b85a2a9e13bc2a0d7bc0f.png)
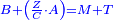
![\scriptstyle{\color{blue}{A+\left[\left(\frac{G}{D}+\frac{1}{H}\right)\sdot\left(K+B\right)\right]=B+\left[\frac{Z}{C}\sdot\left(A+K\right)\right]}}](/mediawiki/images/math/0/b/9/0b902919c6b5bc37321470b17ead7f20.png)
![\scriptstyle{\color{blue}{A+\left[\left(\frac{G}{D}+\frac{1}{H}\right)\sdot\left(K+B\right)\right]=M+L+T}}](/mediawiki/images/math/d/8/7/d8704b61e4e4a6b5557eea3852fc7802.png)
![\scriptstyle{\color{blue}{B+\left[\frac{Z}{C}\sdot\left(A+K\right)\right]=M+L+T}}](/mediawiki/images/math/9/7/7/977e43ddf19671135f696aecef945d0f.png)
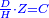
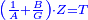
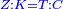
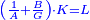
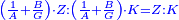
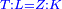
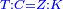
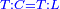
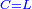
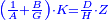



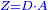
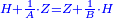
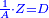


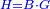
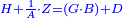
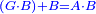
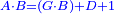


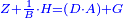

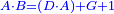

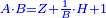

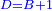
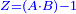


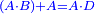
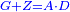
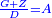

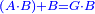
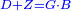
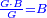
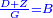
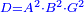
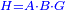
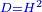
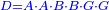

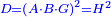
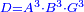
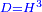
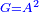
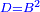
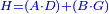
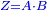

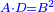

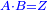
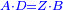
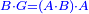
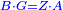
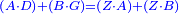
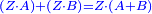

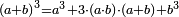

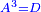
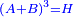

![\scriptstyle{\color{blue}{H-D=3\sdot\left[G\sdot\left(A+B\right)\right]+Z}}](/mediawiki/images/math/b/0/9/b09f392d0b438487a6decbdbf3cd9334.png)
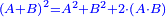

![\scriptstyle{\color{blue}{\left[A^2+B^2+2G\right]\sdot\left(A+B\right)=H}}](/mediawiki/images/math/f/1/b/f1b80fff2f5e7aa1ba4eeb6e41b024d6.png)
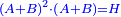
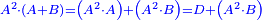

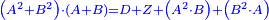

![\scriptstyle{\color{blue}{\left(A^2+B^2\right)\sdot\left(A+B\right)=D+Z+\left[G\sdot\left(A+B\right)\right]}}](/mediawiki/images/math/c/a/4/ca44cc111c2cb95a878f3db960f1c661.png)
![\scriptstyle{\color{blue}{\left[A^2+B^2+2G\right]\sdot\left(A+B\right)=D+Z+3\sdot\left[G\sdot\left(A+B\right)\right]}}](/mediawiki/images/math/f/5/6/f5674833d23608b428ac38048ce2316e.png)
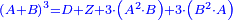
![\scriptstyle{\color{blue}{\left(A+B\right)^3=D+Z+3\sdot\left[G\sdot\left(A+B\right)\right]}}](/mediawiki/images/math/4/f/e/4fead2581c93dc86746257e8302802d8.png)
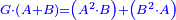
![\scriptstyle{\color{blue}{3\sdot\left[G\sdot\left(A+B\right)\right]=3\sdot\left(A^2\sdot B\right)+3\sdot\left(B^2\sdot A\right)}}](/mediawiki/images/math/c/7/c/c7cb7426772654de2d4e4e1d1fdc994f.png)
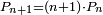
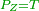

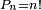
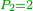
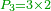
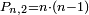


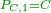
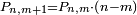

 .
.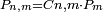

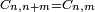
 .
.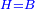 .
. .
.
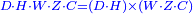
![\scriptstyle{\color{blue}{\left(D\sdot H\right)\times\left[\left(A\sdot B\sdot G\right)+\left(W\sdot Z\sdot C\right)\right]=\left(A\sdot B\sdot G\sdot D\sdot H\right)+\left(D\sdot H\sdot W\sdot Z\sdot C\right)}}](/mediawiki/images/math/4/c/2/4c288561aa62a3d3aecc45ecb32a9f83.png)
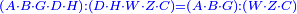
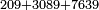

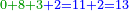
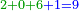
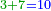

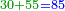

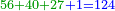
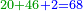
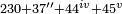
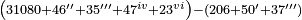











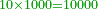

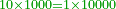
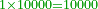
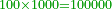


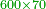
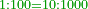


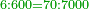

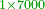
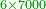
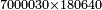
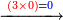
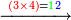
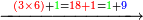


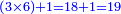
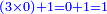



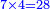
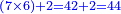
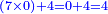



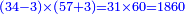

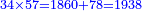

 .
.
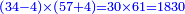


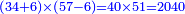

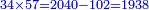
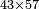
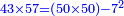
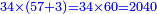
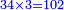
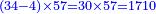
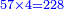
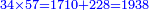
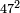
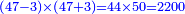


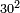
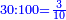

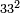
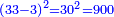
![\scriptstyle{\color{blue}{\left[\left(33-3\right)+33\right]\sdot3=\left(30+33\right)\sdot3=63\sdot3=189}}](/mediawiki/images/math/e/b/b/ebbef80e2418a10a5559b63a02339b1d.png)
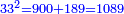
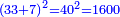
![\scriptstyle{\color{blue}{\left[\left(33+7\right)+33\right]\sdot7=\left(40+33\right)\sdot7=73\sdot7=511}}](/mediawiki/images/math/f/5/6/f56770d8e1bfcb50528a8fcc76c17d91.png)
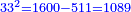

![\scriptstyle{\color{blue}{\begin{align}\scriptstyle33^2&\scriptstyle=\left[\left(\frac{1}{3}\sdot33\right)^2\sdot10\right]-\left(\frac{1}{3}\sdot33\right)^2=\left(11^2\sdot10\right)-11^2=\left(121\sdot10\right)-121\\&\scriptstyle=1210-121=1089\\\end{align}}}](/mediawiki/images/math/f/3/0/f30b8913275ee060c938f5cf6056ffa6.png)
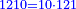
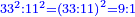
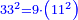
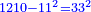
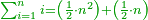
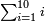
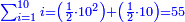
![\scriptstyle{\color{OliveGreen}{\sum_{i=1}^{n} i=n\sdot\left[\frac{1}{2}\sdot\left(n+1\right)\right]}}](/mediawiki/images/math/f/3/9/f393b8be5683e8ce8dd3850bbc6219d9.png)

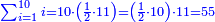
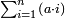
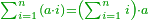


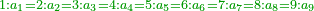

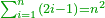
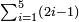
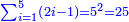

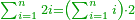


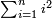
![\scriptstyle{\color{OliveGreen}{\sum_{i=1}^{n} i^2=\left[n-\frac{1}{3}\sdot\left(n-1\right)\right]\sdot\left(\sum_{i=1}^{n} i\right)}}](/mediawiki/images/math/4/9/c/49cf1de561758d7eedb5a24704299738.png)
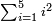
![\scriptstyle{\color{blue}{\sum_{i=1}^{5} i^2=\left[5-\frac{1}{3}\sdot\left(5-1\right)\right]\sdot\left(\sum_{i=1}^{5} i\right)=\left(5-\frac{1}{3}\sdot4\right)\sdot15=\left(4-\frac{1}{3}\right)\sdot15=55}}](/mediawiki/images/math/4/1/c/41c1615e911b365c6346d7a82e1506fe.png)
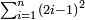
![\scriptstyle{\color{OliveGreen}{\sum_{i=1}^{n} \left(2i-1\right)^2=\left(\sum_{i=1}^{2n} i\right)\sdot\left[\frac{1}{3}\sdot\left(2n-1\right)\right]}}](/mediawiki/images/math/7/1/8/71870b5d7392c0fc87a2344c19633adf.png)

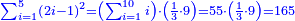
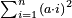

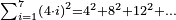
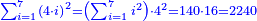
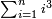
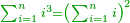

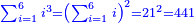
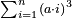

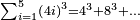
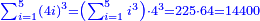

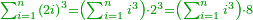
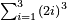
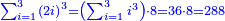
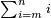

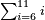
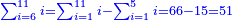
![\scriptstyle a_1\ne d;\;\sum_{i=1}^{n} \left[a_1+d\sdot\left(i-1\right)\right]](/mediawiki/images/math/8/b/e/8bedf010becce9817e1c56f1fb5f1b7c.png)
![\scriptstyle{\color{OliveGreen}{a_1>d\longrightarrow\sum_{i=1}^{n} \left[a_1+d\sdot\left(i-1\right)\right]=d\sdot\sum_{i=1}^{n} i+\left(a_1-d\right)\sdot n}}](/mediawiki/images/math/2/1/8/218302412cca228f52bad14a2746c38f.png)
![\scriptstyle{\color{OliveGreen}{a_1<d\longrightarrow\sum_{i=1}^{n} \left[a_1+d\sdot\left(i-1\right)\right]=d\sdot\sum_{i=1}^{n} i-\left(d-a_1\right)\sdot n}}](/mediawiki/images/math/0/2/2/022ff25de63a6e00ac86b9dc4821e275.png)
![\scriptstyle\sum_{i=1}^{7} \left[\left(3-2\right)+3\sdot\left(i-1\right)\right]](/mediawiki/images/math/3/d/4/3d4323d04d3791acf03e97e731645fc9.png)
![\scriptstyle\sum_{i=1}^{7} \left[\left(3+2\right)+3\sdot\left(i-1\right)\right]](/mediawiki/images/math/f/5/a/f5a17d6574a3481d49667676a62bf189.png)
![\scriptstyle{\color{blue}{\sum_{i=1}^{7} \left[\left(3-2\right)+3\sdot\left(i-1\right)\right]=3\sdot\sum_{i=1}^{7} i-\left(3-1\right)\sdot7=\left(3\sdot28\right)-\left(2\sdot7\right)=84-14=70}}](/mediawiki/images/math/3/6/5/365bc0436e7be35c4725f9bbad64fc5f.png)
![\scriptstyle{\color{blue}{\sum_{i=1}^{7} \left[\left[\left(3-2\right)+3\sdot\left(i-1\right)\right]+\left(3-1\right)\right]=\sum_{i=1}^{7} 3i}}](/mediawiki/images/math/6/9/c/69c6135ca3d98b6729850fd28c4ddbce.png)
![\scriptstyle{\color{blue}{\sum_{i=1}^{7} \left[\left(3+2\right)+3\sdot\left(i-1\right)\right]=3\sdot\sum_{i=1}^{7} i-\left(5-3\right)\sdot7=\left(3\sdot28\right)+\left(2\sdot7\right)=84+14=98}}](/mediawiki/images/math/4/b/e/4beefe0266d6e8dc7f4453a86a2484c8.png)
![\scriptstyle{\color{blue}{\sum_{i=1}^{7} \left[\left[\left(3+2\right)+3\sdot\left(i-1\right)\right]-\left(5-3\right)\right]=\sum_{i=1}^{7} 3i}}](/mediawiki/images/math/f/1/a/f1ad6b98075e7b929cd403c3f63e0098.png)
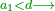
![\scriptstyle{\color{OliveGreen}{\sum_{i=1}^{n} \left[a_1+d\sdot\left(i-1\right)\right]^2=\left[\left(\sum_{i=1}^{n} i^2\right)\sdot d^2\right]-\left[\left[\left(d-a_1\right)\sdot2\sdot\sum_{i=1}^{n} \left[a_1+d\sdot\left(i-1\right)\right]\right]+\left(d-a_1\right)^2\sdot n\right]}}](/mediawiki/images/math/2/1/3/2136708cb3262f5bd52fcf5b5e49013f.png)
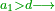
![\scriptstyle{\color{OliveGreen}{\sum_{i=1}^{n} \left[a_1+d\sdot\left(i-1\right)\right]^2=\left[\left(\sum_{i=1}^{n} i^2\right)\sdot d^2\right]+\left[\left[\left(a_1-d\right)\sdot2\sdot\sum_{i=1}^{n} \left[a_1+d\sdot\left(i-1\right)\right]\right]-\left(a_1-d\right)^2\sdot n\right]}}](/mediawiki/images/math/3/4/1/341e85aad0358efa97dd849dee5686b4.png)
![\scriptstyle\sum_{i=1}^{7} \left[\left(3+2\right)+3\sdot\left(i-1\right)\right]^2](/mediawiki/images/math/8/7/c/87c7611296c812bbd617dfdf8053e0d6.png)
![\scriptstyle\sum_{i=1}^{7} \left[\left(3-2\right)+3\sdot\left(i-1\right)\right]^2](/mediawiki/images/math/9/3/9/9395f79f2c22eb247afb676ba9ac9b42.png)
![\scriptstyle{\color{blue}{\left[\left(5-3\right)\sdot2\sdot\sum_{i=1}^{7} \left[5+3\sdot\left(i-1\right)\right]\right]-\left(5-3\right)^2\sdot 7=\left(2\sdot2\sdot98\right)-2^2\sdot 7=\left(2\sdot196\right)-4\sdot 7=392-28=364}}](/mediawiki/images/math/0/0/3/0035541a558896c31483b5eefb433946.png)
![\scriptstyle{\color{blue}{\left[\left(5-3\right)\sdot2\sdot\sum_{i=1}^{7} \left[3+3\sdot\left(i-1\right)\right]\right]+\left(5-3\right)^2\sdot 7=\left(2\sdot2\sdot84\right)+2^2\sdot 7=336+4\sdot 7=336+28=364}}](/mediawiki/images/math/a/d/b/adbee6d1927099d40e0f2dd11a458cb5.png)
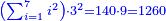
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle\sum_{i=1}^{7} \left[\left(3+2\right)+3\sdot\left(i-1\right)\right]^2&\scriptstyle=\left[\left(\sum_{i=1}^{7} i^2\right)\sdot3^2\right]+\left[\left[\left(5-3\right)\sdot2\sdot\sum_{i=1}^{7} \left[5+3\sdot\left(i-1\right)\right]\right]-\left(5-3\right)^2\sdot 7\right]\\&\scriptstyle=1260+364=1624\\\end{align}}}](/mediawiki/images/math/7/7/f/77f8441d46ca6150e06b29a9e6504401.png)
![\scriptstyle{\color{blue}{\left[\left(3-1\right)\sdot2\sdot\sum_{i=1}^{7} \left[1+3\sdot\left(i-1\right)\right]\right]+\left(3-1\right)^2\sdot 7=\left(2\sdot2\sdot70\right)+2^2\sdot 7=\left(2\sdot140\right)+4\sdot 7=280+28=308}}](/mediawiki/images/math/0/e/1/0e14c827db5d1eead6906c83ae01e699.png)
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle\sum_{i=1}^{7} \left[\left(3-2\right)+3\sdot\left(i-1\right)\right]^2&\scriptstyle=\left[\left(\sum_{i=1}^{7} i^2\right)\sdot3^2\right]-\left[\left[\left(3-1\right)\sdot2\sdot\sum_{i=1}^{7} \left[1+3\sdot\left(i-1\right)\right]\right]+\left(3-1\right)^2\sdot 7\right]\\&\scriptstyle=1260-308=953\\\end{align}}}](/mediawiki/images/math/d/f/b/dfb01970faf0feb2f4fe02c8f265d5f1.png)
![\scriptstyle{\color{blue}{\sum_{i=1}^{7} \left[\left[\left(3-2\right)+3\sdot\left(i-1\right)\right]^2+\left(3-1\right)\right]}}](/mediawiki/images/math/e/d/e/edef43e151df2ac9d529ce69abef60cd.png)
![\scriptstyle{\color{blue}{\left[\left(3-2\right)+3\sdot\left(i-1\right)+2\right]^2-\left[\left(3-2\right)+3\sdot\left(i-1\right)\right]^2=2\sdot2\sdot\left[\left(3-2\right)+3\sdot\left(i-1\right)\right]+2^2}}](/mediawiki/images/math/2/6/3/2634573486a01e41a859f4d75dd74e69.png)
![\scriptstyle{\color{OliveGreen}{\begin{align}\scriptstyle\sum_{i=1}^{n} \left[a_1+d\sdot\left(i-1\right)\right]^3&\scriptstyle=\left[\left(\sum_{i=1}^{n} i^3\right)\sdot d^3\right]+\left[3\sdot\left(d-a_1\right)\sdot\sum_{i=1}^{n} \left[a_1+d\sdot\left(i-1\right)\right]^2\right]\\&\scriptstyle+\left[3\sdot\left(d-a_1\right)^2\sdot\sum_{i=1}^{n} \left[a_1+d\sdot\left(i-1\right)\right]\right]+\left(d-a_1\right)^3\sdot n\\\end{align}}}](/mediawiki/images/math/7/e/9/7e923e182c591219e7519e81c93dfd84.png)
![\scriptstyle\sum_{i=1}^{7} \left[\left(3-2\right)+3\sdot\left(i-1\right)\right]^3](/mediawiki/images/math/a/a/9/aa9987f51ea6172302bf409347a1e138.png)
![\scriptstyle{\color{blue}{3\sdot\left(3-1\right)\sdot\sum_{i=1}^{7} \left[1+3\sdot\left(i-1\right)\right]^2=3\sdot2\sdot952=6\sdot952=5712}}](/mediawiki/images/math/e/2/2/e22c3464e179ee22e721fa6dc4baa6c1.png)
![\scriptstyle{\color{blue}{3\sdot\left(3-1\right)^2\sdot\sum_{i=1}^{7} \left[1+3\sdot\left(i-1\right)\right]=3\sdot2^2\sdot70=12\sdot70=840}}](/mediawiki/images/math/0/6/8/06868efcd306a07ab76546ac647ca2a3.png)
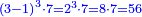
![\scriptstyle{\color{blue}{\begin{align}&\scriptstyle\left[3\sdot\left(3-1\right)\sdot\sum_{i=1}^{7} \left[1+3\sdot\left(i-1\right)\right]^2\right]+\left[3\sdot\left(3-1\right)^2\sdot\sum_{i=1}^{7} \left[1+3\sdot\left(i-1\right)\right]\right]+\left(3-1\right)^3\sdot7\\&\scriptstyle=5712+840+56=6608\\\end{align}}}](/mediawiki/images/math/5/4/8/5488d78a654ff66b70ce28474c4a3436.png)

![\scriptstyle\sum_{i=1}^{7} \left[\left(3+2\right)+3\sdot\left(i-1\right)\right]^3](/mediawiki/images/math/e/a/3/ea347b2b565feb93cf85232915d6b23b.png)
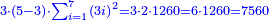
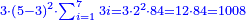
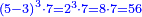
![\scriptstyle{\color{blue}{\begin{align}&\scriptstyle\left[3\sdot\left(5-3\right)\sdot\sum_{i=1}^{7} \left(3i\right)^2\right]+\left[3\sdot\left(5-3\right)^2\sdot\sum_{i=1}^{7} 3i\right]+\left(5-3\right)^3\sdot7\\&\scriptstyle=7560+1008+56=8624\\\end{align}}}](/mediawiki/images/math/6/7/5/6750f355ffc3f53722fcc1b8fe240003.png)
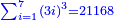
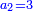
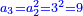
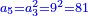
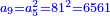
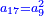
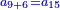

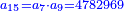


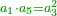
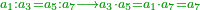

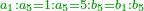
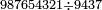


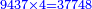
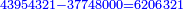
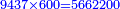

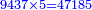
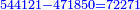


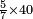

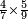
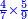
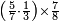
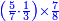
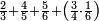
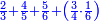
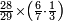
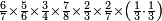
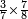
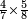
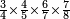
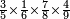
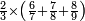
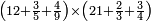
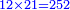
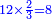
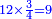
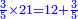
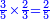

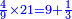
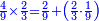

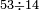
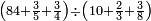
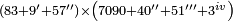
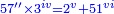
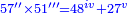
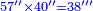
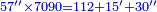
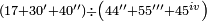


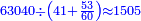
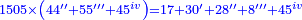
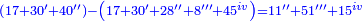
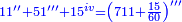
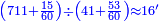
![\scriptstyle{\color{blue}{\left(11^{\prime\prime}+51^{\prime\prime\prime}+15^{iv}\right)-\left[16^\prime\times\left(44^{\prime\prime}+55^{\prime\prime\prime}+45^{iv}\right)\right]=41^{\prime\prime\prime}+11^{iv}}}](/mediawiki/images/math/e/3/6/e36a5d98432d73a358ebf4d13e9e9349.png)
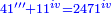
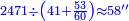
![\scriptstyle{\color{blue}{\left(41^{\prime\prime\prime}+11^{iv}\right)-\left[58^{\prime\prime}\times\left(44^{\prime\prime}+55^{\prime\prime\prime}+45^{iv}\right)\right]=30^{\prime\prime\prime}+42^{iv}}}](/mediawiki/images/math/c/5/5/c559e2b52de2b8e327e5fbee40204e4d.png)
![\scriptstyle{\color{blue}{\left(30^{\prime\prime\prime}+42^{iv}\right)-\left[1^{\prime\prime}\times\left(44^{\prime\prime}+55^{\prime\prime\prime}+45^{iv}\right)\right]=7^v+45^{vi}}}](/mediawiki/images/math/a/0/8/a08212e9f4a027a15135fe8b28a0078e.png)

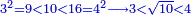
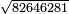
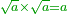
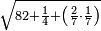
![\scriptstyle{\color{blue}{\sqrt{82+\frac{1}{4}+\left(\frac{2}{7}\sdot\frac{1}{7}\right)}=\frac{\sqrt{\left[82+\frac{1}{4}+\left(\frac{2}{7}\sdot\frac{1}{7}\right)\right]\sdot196}}{\sqrt{196}}=\frac{\sqrt{16129}}{\sqrt{196}}=\frac{127}{14}=9+\left(\frac{1}{2}\sdot\frac{1}{7}\right)}}](/mediawiki/images/math/e/d/4/ed4b027c6ebf52aae7b0e477c5c71a4f.png)
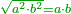
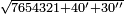

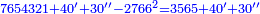

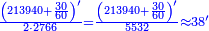
![\scriptstyle{\color{blue}{\left(3565+40^{\prime}+30^{\prime\prime}\right)-\left[38^{\prime}\sdot\left[\left(2\sdot2766\right)+38^{\prime}\right]\right]=61+40^{\prime}+26^{\prime\prime}=3700^{\prime}+26^{\prime\prime}}}](/mediawiki/images/math/2/9/b/29b4f88d520018f7b263d08a15f9f742.png)

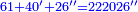

![\scriptstyle{\color{blue}{\begin{align}\scriptstyle\left(61+40^{\prime}+26^{\prime\prime}\right)-\left[40^{\prime\prime}\sdot\left[\left[2\sdot\left(2766+38^{\prime}\right)\right]+40^{\prime\prime}\right]\right]&\scriptstyle=11^{\prime}+34^{\prime\prime}+53^{\prime\prime\prime}+20^{iv}\\&\scriptstyle=694^{\prime\prime}+53^{\prime\prime\prime}+20^{\prime\prime\prime\prime}\\\end{align}}}](/mediawiki/images/math/1/a/2/1a2fc1ce2151c958c003188aa94b71d8.png)


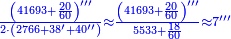
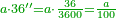


![\scriptstyle{\color{blue}{\begin{align}\scriptstyle\frac{987543+12^\prime+36^{\prime\prime}}{100}&\scriptstyle=9875+\frac{43+12^\prime+36^{\prime\prime}}{100}=9875+\left[\left(43+12^\prime+36^{\prime\prime}\right)\sdot36^{\prime\prime}\right]\\&\scriptstyle=9875+25^\prime+55^{\prime\prime}+33^{\prime\prime\prime}+36^{iv}\\\end{align}}}](/mediawiki/images/math/8/d/a/8da67fb66ab840830a793e3b62b3463b.png)
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle\frac{9875+25^\prime+55^{\prime\prime}+33^{\prime\prime\prime}+36^{iv}}{100}&\scriptstyle=98+\frac{75+25^\prime+55^{\prime\prime}+33^{\prime\prime\prime}+36^{iv}}{100}\\&\scriptstyle=98+\left[\left(75+25^\prime+55^{\prime\prime}+33^{\prime\prime\prime}+36^{iv}\right)\sdot36^{\prime\prime}\right]\\&\scriptstyle=98+45^\prime+15^{\prime\prime}+33^{\prime\prime\prime}+20^{iv}+9^v+36^{vi}<100\\\end{align}}}](/mediawiki/images/math/6/f/9/6f995965609acf6a5f352940d5527a10.png)
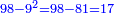
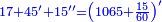
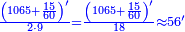
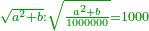
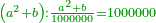
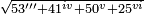
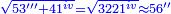
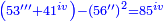
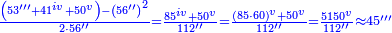
![\scriptstyle{\color{blue}{\left(85^{iv}+50^{v}+25^{vi}\right)-\left[45^{\prime\prime\prime}\sdot\left(2\sdot56^{\prime\prime}+45^{\prime\prime\prime}\right)\right]=76^{v}+40^{vi}}}](/mediawiki/images/math/2/f/7/2f7c550c200add7bdb1f98fdffde5df5.png)
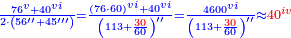
![\scriptstyle\sqrt[3]{654321}](/mediawiki/images/math/5/d/e/5ded0e79faf759037229fdfbfa52feb2.png)
![\scriptstyle{\color{blue}{\sqrt[3]{512}=8}}](/mediawiki/images/math/d/3/6/d3684b29eb0adcf365b12d48df153a7a.png)
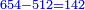
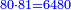

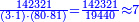
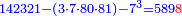
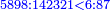

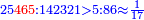

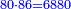

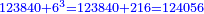
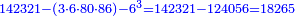
![\scriptstyle\sqrt[3]{5987654321}](/mediawiki/images/math/1/5/4/154d7bdef195b69661f20d89a1a34085.png)
![\scriptstyle\sqrt[3]{44+\left(\frac{5}{7}\sdot\frac{1}{4}\right)+\left(\frac{13}{7}\sdot\frac{1}{7}\sdot\frac{1}{4}\sdot\frac{1}{4}\right)+\left(\frac{27}{7}\sdot\frac{1}{7}\sdot\frac{1}{7}\sdot\frac{1}{4}\sdot\frac{1}{4}\sdot\frac{1}{4}\right)}](/mediawiki/images/math/c/4/c/c4c390f780300248459e24576307674a.png)
![\scriptstyle{\color{blue}{\begin{align}&\scriptstyle\sqrt[3]{44+\left(\frac{5}{7}\sdot\frac{1}{4}\right)+\left(\frac{13}{7}\sdot\frac{1}{7}\sdot\frac{1}{4}\sdot\frac{1}{4}\right)+\left(\frac{27}{7}\sdot\frac{1}{7}\sdot\frac{1}{7}\sdot\frac{1}{4}\sdot\frac{1}{4}\sdot\frac{1}{4}\right)}\\&\scriptstyle=\frac{\sqrt[3]{\left[44+\left(\frac{5}{7}\sdot\frac{1}{4}\right)+\left(\frac{13}{7}\sdot\frac{1}{7}\sdot\frac{1}{4}\sdot\frac{1}{4}\right)+\left(\frac{27}{7}\sdot\frac{1}{7}\sdot\frac{1}{7}\sdot\frac{1}{4}\sdot\frac{1}{4}\sdot\frac{1}{4}\right)\right]\sdot\left(7^3\sdot4^3\right)}}{\sqrt[3]{7^3\sdot4^3}}\\&\scriptstyle=\frac{99}{28}=3+\frac{15}{28}\\\end{align}}}](/mediawiki/images/math/b/9/6/b96e4c0c4fbf3d23dad729e5ba4f8ade.png)
![\scriptstyle\sqrt[3]{59^\prime+23^{\prime\prime}+7^{\prime\prime\prime}+40^{iv}}](/mediawiki/images/math/2/a/f/2afe94cbcd84632c5bbc55c3c454d878.png)
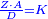

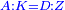

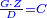
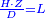

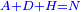
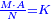
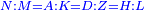

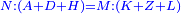



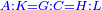
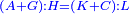
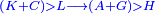



![\scriptstyle{\color{blue}{S:H=\left[\left(K+C\right)-L\right]:L}}](/mediawiki/images/math/0/b/9/0b9c4afcb1be9f2d8927bff0541458db.png)
![\scriptstyle{\color{blue}{S:\left[\left(K+C\right)-L\right]=H:L}}](/mediawiki/images/math/2/7/6/27613ce95f84f90d8647e9e9ec25b914.png)



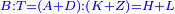

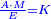
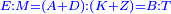
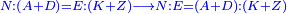
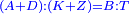

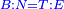


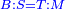

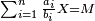
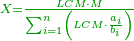

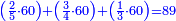
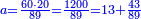



![\scriptstyle{\color{blue}{\left[\left(\frac{2}{5}\sdot60\right)+\left(\frac{3}{4}\sdot60\right)+\left(\frac{1}{3}\sdot60\right)\right]:60=\left(\frac{2}{5}a+\frac{3}{4}a+\frac{1}{3}a\right):a}}](/mediawiki/images/math/0/8/e/08eea7aa880ebf29610ef50897993f71.png)
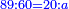
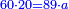
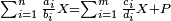
![\scriptstyle{\color{OliveGreen}{X=\frac{LCM\sdot P}{\left[\sum_{i=1}^n \left(LCM\sdot\frac{a_i}{b_i}\right)\right]-\left[\sum_{i=1}^m \left(LCM\sdot\frac{c_i}{d_i}\right)\right]}}}](/mediawiki/images/math/c/5/4/c540179442b53d7ceee089f009d06ba4.png)
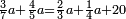
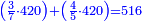



![\scriptstyle{\color{blue}{\left[\left(\frac{3}{7}\sdot420\right)+\left(\frac{4}{5}\sdot420\right)\right]:420=\left(\frac{3}{7}a+\frac{4}{5}a\right):a}}](/mediawiki/images/math/6/a/9/6a9267fa3e8781049316dcfd65d857f2.png)
![\scriptstyle{\color{blue}{\left[\left(\frac{3}{7}\sdot420\right)+\left(\frac{4}{5}\sdot420\right)\right]:\left(\frac{3}{7}a+\frac{4}{5}a\right)=420:a}}](/mediawiki/images/math/a/9/1/a915ae6062576d141b22b0c66f3ffe28.png)
![\scriptstyle{\color{blue}{\left[\left(\frac{2}{3}\sdot420\right)+\left(\frac{1}{4}\sdot420\right)\right]:\left(\frac{2}{3}a+\frac{1}{4}a\right)=420:a}}](/mediawiki/images/math/5/0/0/500b4171ec6647ccf418ed41181d50ef.png)
![\scriptstyle{\color{blue}{\left[\left(\frac{3}{7}\sdot420\right)+\left(\frac{4}{5}\sdot420\right)\right]:\left(\frac{3}{7}a+\frac{4}{5}a\right)=\left[\left(\frac{2}{3}\sdot420\right)+\left(\frac{1}{4}\sdot420\right)\right]:\left(\frac{2}{3}a+\frac{1}{4}a\right)}}](/mediawiki/images/math/d/8/4/d848454f8c9f9644b6b687c796b2e0d6.png)
![\scriptstyle{\color{blue}{\left[\left(\frac{3}{7}\sdot420\right)+\left(\frac{4}{5}\sdot420\right)\right]:\left[\left(\frac{2}{3}\sdot420\right)+\left(\frac{1}{4}\sdot420\right)\right]=\left(\frac{3}{7}a+\frac{4}{5}a\right):\left(\frac{2}{3}a+\frac{1}{4}a\right)}}](/mediawiki/images/math/8/b/6/8b636870277c957d3d8b58a71991ab3a.png)
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle\left[\left(\frac{3}{7}\sdot420\right)+\left(\frac{4}{5}\sdot420\right)\right]&\scriptstyle:\left[\left[\left(\frac{3}{7}\sdot420\right)+\left(\frac{4}{5}\sdot420\right)\right]-\left[\left(\frac{2}{3}\sdot420\right)+\left(\frac{1}{4}\sdot420\right)\right]\right]\\&\scriptstyle=\left(\frac{3}{7}a+\frac{4}{5}a\right):\left[\left(\frac{3}{7}a+\frac{4}{5}a\right)-\left(\frac{2}{3}a+\frac{1}{4}a\right)\right]\\\end{align}}}](/mediawiki/images/math/b/1/e/b1e3b1188550bdb0e3e5efbec2452b09.png)
![\scriptstyle{\color{blue}{\left[\left(\frac{3}{7}\sdot420\right)+\left(\frac{4}{5}\sdot420\right)\right]:131=\left(\frac{3}{7}a+\frac{4}{5}a\right):20}}](/mediawiki/images/math/c/a/b/cab06e4b9806531899d40008ed0429a7.png)
![\scriptstyle{\color{blue}{\left[\left(\frac{3}{7}\sdot420\right)+\left(\frac{4}{5}\sdot420\right)\right]:\left(\frac{3}{7}a+\frac{4}{5}a\right)=131:20}}](/mediawiki/images/math/7/8/5/785f7d4e359dca94b959cfe9ad620c75.png)
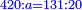

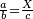
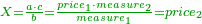
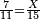
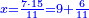



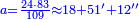

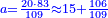


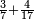
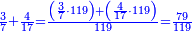
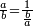
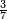
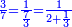
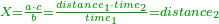
![\scriptstyle\left(13\sdot24\right):\left(7+36'+57''\right)=\left[\left(3\sdot24\right)+17+52'+16''\right]:a](/mediawiki/images/math/7/a/a/7aafb9e9d321ca8d15edcdaa17f0c657.png)
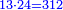

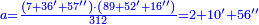
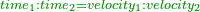
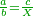
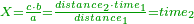
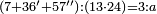
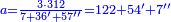

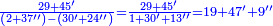
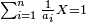
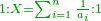
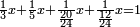
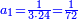
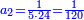

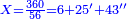
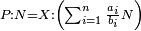
![\scriptstyle{\color{OliveGreen}{X=\frac{\left[\sum_{i=1}^n \left[\left(LCM\right)\sdot\frac{a_i}{b_i}\right]\right]\sdot\frac{P}{N}\sdot N}{LCM}}}](/mediawiki/images/math/7/b/3/7b3428abdb4dcb08bda2b4a6106d7e56.png)
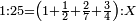
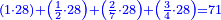
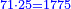
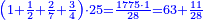
![\scriptstyle{\color{OliveGreen}{\left[\sum_{i=1}^n \left[\left(LCM\right)\sdot\frac{a_i}{b_i}\right]\right]:\left[\sum_{i=1}^n \left(1\sdot\frac{a_i}{b_i}\right)\right]=\left(LCM\right):1}}](/mediawiki/images/math/a/4/2/a42a0462aa2e99ddbd531b8a42d7d34d.png)
![\scriptstyle{\color{OliveGreen}{price_{\left[\sum_{i=1}^n \left[\left(LCM\right)\sdot\frac{a_i}{b_i}\right]\right]}:price_{\left[\sum_{i=1}^n \left(1\sdot\frac{a_i}{b_i}\right)\right]}=\left(LCM\right):1}}](/mediawiki/images/math/d/d/2/dd263e80acf2ebaa8806ac99e6038291.png)
![\scriptstyle{\color{OliveGreen}{price_{\left[\sum_{i=1}^n \left[\left(LCM\right)\sdot\frac{a_i}{b_i}\right]\right]}\sdot1=price_{\left[\sum_{i=1}^n \left(1\sdot\frac{a_i}{b_i}\right)\right]}\sdot\left(LCM\right)}}](/mediawiki/images/math/b/6/d/b6d68a29ab1545e8b989ab710a0e3436.png)
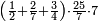
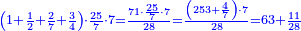

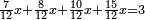
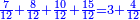
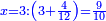

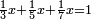
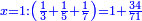
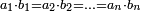
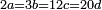




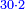

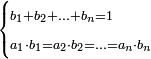
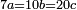
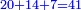
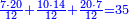
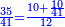
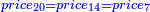
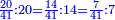
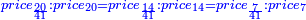
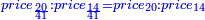
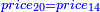
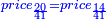
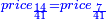
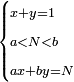
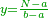
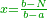
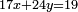

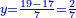
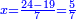
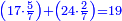
![\scriptstyle{\color{blue}{\left[17\sdot\left(24-19\right)\right]+\left[24\sdot\left(19-17\right)\right]=\left(17\sdot5\right)+\left(24\sdot2\right)=19\sdot7=19\sdot\left(24-17\right)}}](/mediawiki/images/math/6/1/4/614db8257c79374611ae4fae7cee0b50.png)
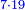
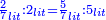

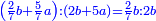
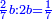
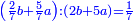
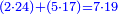
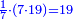
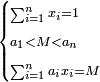
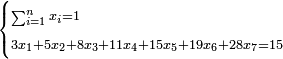
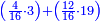
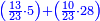
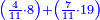
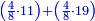
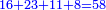
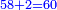
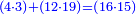
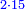
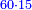
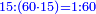
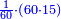

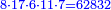


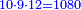
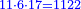
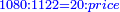


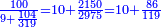
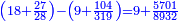
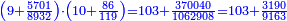


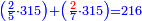
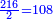
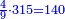
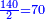

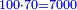
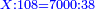


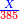


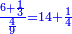
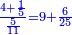
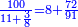
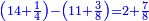
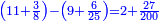
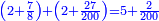
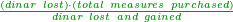

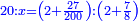

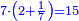

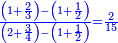
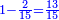
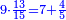

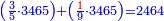


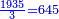
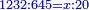
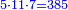
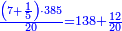
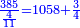

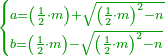
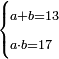
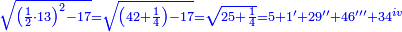
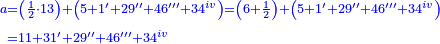
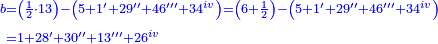

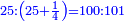

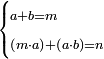
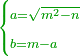
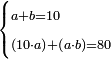
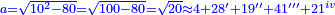
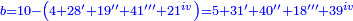

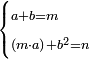
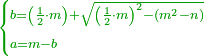
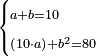
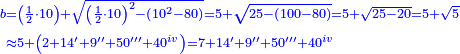
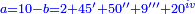
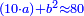
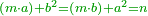
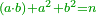

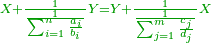

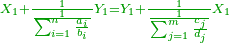
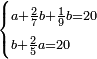
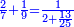
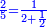
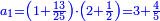
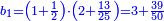

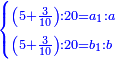
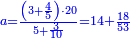
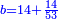


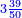
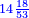
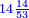

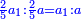
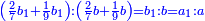

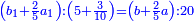
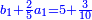
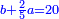
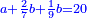

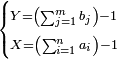
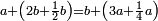
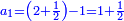
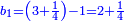
![\scriptstyle{\color{blue}{a_1+\left(2b_1+\frac{1}{2}b_1\right)=\left(a_1+b_1\right)+\left[\left(1+\frac{1}{2}\right)\sdot\left(2+\frac{1}{4}\right)\right]=\left(a_1+b_1\right)+\left(a_1\sdot b_1\right)}}](/mediawiki/images/math/4/7/0/4702deb78c486394688f2053b4d02ea8.png)
![\scriptstyle{\color{blue}{b_1+\left(3a_1+\frac{1}{4}a_1\right)=\left(b_1+a_1\right)+\left[\left(2+\frac{1}{4}\right)\sdot\left(1+\frac{1}{2}\right)\right]=\left(b_1+a_1\right)+\left(b_1\sdot a_1\right)}}](/mediawiki/images/math/c/4/7/c4748dea62920f0cebf3947736b27122.png)
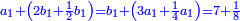
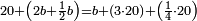
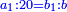

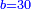
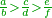

![\scriptstyle{\color{OliveGreen}{\begin{cases}\scriptstyle X=\frac{1}{\frac{e}{f}}+\left(\frac{1}{\frac{c}{d}}-\frac{1}{\frac{a}{b}}\right)+\left[\left(\frac{1}{\frac{a}{b}}-2\right)\sdot\left(\frac{1}{\frac{c}{d}}\sdot\frac{1}{\frac{e}{f}}\right)\right]\\\scriptstyle Y=X+2\sdot\left[\left(\frac{1}{\frac{c}{d}}-\frac{1}{\frac{a}{b}}\right)\sdot\left(\frac{1}{\frac{e}{f}}-1\right)\right]\\\scriptstyle Z=Y+2\sdot\left[\left(\frac{1}{\frac{a}{b}}-1\right)\sdot\left(\frac{1}{\frac{e}{f}}-\frac{1}{\frac{c}{d}}\right)\right]\end{cases}}}](/mediawiki/images/math/1/b/6/1b6204af7e826f12a90c677ea03fcb32.png)
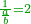
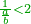
![\scriptstyle{\color{OliveGreen}{X=\frac{1}{\frac{e}{f}}+\left(\frac{1}{\frac{c}{d}}-\frac{1}{\frac{a}{b}}\right)-\left[\left(2-\frac{1}{\frac{a}{b}}\right)\sdot\left(\frac{1}{\frac{c}{d}}\sdot\frac{1}{\frac{e}{f}}\right)\right]}}](/mediawiki/images/math/9/9/5/995eef63abc1c775988e14b6436231af.png)

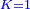

![\scriptstyle{\color{blue}{a_2=N=M+\left[2\sdot\left(H\times T\right)\right]}}](/mediawiki/images/math/1/3/c/13c8faab4949dfdb918669d568dc0d57.png)
![\scriptstyle{\color{blue}{a_3=S=N+\left[2\sdot\left(L\times Z\right)\right]}}](/mediawiki/images/math/a/0/b/a0bbe957559ab338ddc3f8bb7bd99b44.png)
![\scriptstyle{\color{blue}{a_2=N=\left[\left(G+H\right)-\left(D\times B\times G\right)\right]+\left[2\sdot\left(H\times T\right)\right]}}](/mediawiki/images/math/7/e/b/7ebded9b898b629599ce15678ade6df9.png)
![\scriptstyle{\color{blue}{a_3=S=\left[\left(G+H\right)-\left(D\sdot B\sdot G\right)\right]+\left[2\sdot\left(H\sdot T\right)\right]+\left[2\sdot\left(L\sdot Z\right)\right]}}](/mediawiki/images/math/6/3/c/63ce37b2015f2841718ad892d5ac8e29.png)
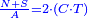
![\scriptstyle{\color{blue}{\frac{1}{2}\sdot\left(N+S\right)=G+H+\left[2\sdot\left(H\times T\right)\right]+\left(L\times Z\right)-\left(D\times B\times G\right)}}](/mediawiki/images/math/2/4/6/246acb8b300e9a489e5357a9fa562035.png)

![\scriptstyle{\color{blue}{\frac{1}{2}\sdot\left(N+S\right)=G+H+\left[2\sdot\left(H\times T\right)\right]+\left(L\times Z\right)+\left[L\sdot\left(C+G\right)\right]-\left[\left(D\times B\times G\right)+\left[L\sdot\left(C+G\right)\right]\right]}}](/mediawiki/images/math/4/4/5/44533c48e547f708c04c1e7731bab22c.png)
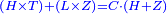



![\scriptstyle{\color{blue}{\left[C\sdot\left(H+Z\right)\right]+\left(C\times L\right)=C\times T}}](/mediawiki/images/math/4/8/4/4849ebf6c8dff353344c0eb3d3c738c5.png)
![\scriptstyle{\color{blue}{\left[L\sdot\left(C+G\right)\right]-\left(L\times C\right)=L\times G}}](/mediawiki/images/math/7/d/7/7d7eb7007300b2229bc086df3cbc71e5.png)
![\scriptstyle{\color{blue}{G+H+\left(H\times T\right)-\left[\left(D\times B\times G\right)+\left[L\sdot\left(C+G\right)\right]\right]=\left(L\times C\times T\right)}}](/mediawiki/images/math/7/9/5/79568effb17cbaca27f0f07cd83afc20.png)
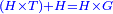


![\scriptstyle{\color{blue}{\left[\left(D+L\right)\sdot\left(B\times G\right)\right]-\left(D\times B\times G\right)=\left(L\times B\times G\right)}}](/mediawiki/images/math/6/0/4/6040c81203cff0778a5cc43ad655c571.png)

![\scriptstyle{\color{blue}{\left[\left(L\times C\right)\sdot T\right]+\left(L\times C\right)=\left(L\times C\times G\right)}}](/mediawiki/images/math/b/2/f/b2fea0919588f68cb6c1189b7255a240.png)
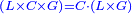

![\scriptstyle{\color{blue}{\left(L\times B\times G\right)-\left[L\sdot\left(C+G\right)\right]=\left(L\times C\times T\right)}}](/mediawiki/images/math/1/5/6/156c1b39dd7912628df6fa5558d1d937.png)
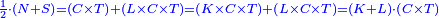
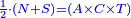
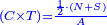
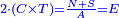
![\scriptstyle{\color{blue}{\frac{1}{2}\sdot\left(M+S\right)=H+G+\left(H\times T\right)+\left(L\times Z\right)+\left(L\times B\right)-\left[\left(D\times B\times G\right)+\left(L\times B\right)\right]}}](/mediawiki/images/math/d/1/d/d1de3f1c0b24976731334b91185eb794.png)
![\scriptstyle{\color{blue}{H+G+\left(H\times T\right)+\left(L\times Z\right)+\left(L\times B\right)-\left[\left(D\times B\times G\right)+\left(L\times B\right)\right]=\left(L\sdot B\times T\right)}}](/mediawiki/images/math/a/2/d/a2d0e931d062de33d350fe28089f06ac.png)
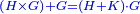
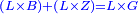

![\scriptstyle{\color{blue}{\left[\left(H+K\right)\sdot G\right]+\left(L\times G\right)=\left(L+D\right)\sdot\left(B\times G\right)}}](/mediawiki/images/math/2/5/8/25872aa23e98994080fe2981ab73c44f.png)
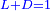


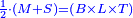
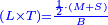
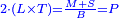
![\scriptstyle{\color{blue}{\frac{1}{2}\sdot\left(M+N\right)=H+G+\left(H\times T\right)+\left(L\times G\right)-\left[\left(D\times B\times G\right)+\left(L\times G\right)\right]}}](/mediawiki/images/math/a/4/e/a4e12f0e8dbdc4655ec2ba157118a5bb.png)
![\scriptstyle{\color{blue}{H+G+\left(H\times T\right)+\left(L\times G\right)-\left[\left(D\times B\times G\right)+\left(L\times G\right)\right]=\left(L\times C\times G\right)}}](/mediawiki/images/math/1/e/7/1e72bc45bcccca76f28d4a47563dcdcc.png)
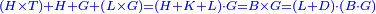
![\scriptstyle{\color{blue}{\left[\left(L+D\right)\sdot\left(B\times G\right)\right]-\left(D\times B\times G\right)=\left(L\times B\times G\right)=B\sdot\left(L\times G\right)}}](/mediawiki/images/math/e/6/7/e67fbb6dcee6ec0e4bd3e54690c9b592.png)
![\scriptstyle{\color{blue}{\left[B\sdot\left(L\times G\right)\right]-\left(L\times G\right)=C\sdot\left(L\times G\right)=\left(L\times C\times G\right)=G\sdot\left(L\times C\right)}}](/mediawiki/images/math/e/f/4/ef415d7d63793034211b35bec1fd46ec.png)
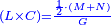

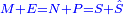
![\scriptstyle{\color{blue}{M+E=M+\left[2\sdot\left(C\times T\right)\right]}}](/mediawiki/images/math/9/a/b/9ab8c4e33dce562a80e4bcba2f57faec.png)
![\scriptstyle{\color{blue}{N+P=M+\left[2\sdot\left(H\times T\right)\right]+\left[2\sdot\left(L\times T\right)\right]}}](/mediawiki/images/math/d/3/6/d36554bc7edd1a6376f5a482db2b05d0.png)
![\scriptstyle{\color{blue}{N+P=M+\left[2\sdot\left(C\times T\right)\right]}}](/mediawiki/images/math/4/3/4/43455fcadda0e877daeca771b3b26634.png)
![\scriptstyle{\color{blue}{S+\hat S=M+\left[2\sdot\left(H\times T\right)\right]+\left[2\sdot\left(Z\times L\right)\right]+\left[2\sdot\left(C\times L\right)\right]}}](/mediawiki/images/math/e/6/f/e6f14b8c7982ac8ec136a7cf84c04fa1.png)
![\scriptstyle{\color{blue}{\left[2\sdot\left(Z\times L\right)\right]+\left[2\sdot\left(C\times L\right)\right]=\left[2\sdot\left[\left(Z+C\right)\sdot L\right]\right]=2\sdot\left(T\times L\right)}}](/mediawiki/images/math/6/e/f/6ef35fa8ec2db3326171e74464f21570.png)
![\scriptstyle{\color{blue}{\left[2\sdot\left(T\times L\right)\right]+\left[2\sdot\left(T\times H\right)\right]=2\sdot\left[T\sdot\left(L+H\right)\right]}}](/mediawiki/images/math/d/7/0/d702c5a932d1c140ed73b40f0c220103.png)
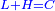
![\scriptstyle{\color{blue}{2\sdot\left[T\sdot\left(L+H\right)\right]=2\sdot\left(T\times C\right)}}](/mediawiki/images/math/6/4/3/643c78819eb511b2f6fb9eed995a923e.png)
![\scriptstyle{\color{blue}{S+\hat S=M+\left[2\sdot\left(C\times T\right)\right]}}](/mediawiki/images/math/3/f/c/3fca713858636d3df250334829ed09c6.png)
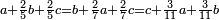
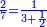
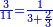
![\scriptstyle{\color{blue}{a=\left(3+\frac{2}{3}\right)+\left[\left(3+\frac{1}{2}\right)-\left(2+\frac{1}{2}\right)\right]+\left[\left[\left(2+\frac{1}{2}\right)-2\right]\sdot\left[\left(3+\frac{1}{2}\right)\sdot\left(3+\frac{2}{3}\right)\right]\right]=11+\frac{1}{12}}}](/mediawiki/images/math/c/f/f/cff44fec8c723d77da824bd8a360b890.png)
![\scriptstyle{\color{blue}{{\color{OliveGreen}{b}}=\left(11+\frac{1}{12}\right)+2\sdot\left[\left[\left(3+\frac{1}{2}\right)-\left(2+\frac{1}{2}\right)\right]\sdot\left[\left(3+\frac{2}{3}\right)-1\right]\right]=16+\frac{{\color{red}{5}}}{12}}}](/mediawiki/images/math/f/3/9/f3921ae6b43e22c226593f7f0fa22d64.png)
![\scriptstyle{\color{blue}{{\color{OliveGreen}{c=\left(16+\frac{5}{12}\right)+2\sdot\left[\left[\left(2+\frac{1}{2}\right)-1\right]\sdot\left[\left(3+\frac{2}{3}\right)-\left(3+\frac{1}{2}\right)\right]\right]}}=16+\frac{11}{12}}}](/mediawiki/images/math/3/d/2/3d28edc4e1415eec00de682a0841e78a.png)
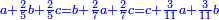
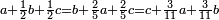
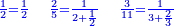
![\scriptstyle{\color{blue}{a=\left(3+\frac{2}{3}\right)+\left[\left(2+\frac{1}{2}\right)-2\right]=4+\frac{1}{6}}}](/mediawiki/images/math/f/b/1/fb1d5cdbe16dabcf73c9bdc309172786.png)
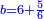

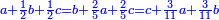
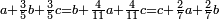
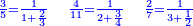
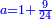
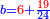

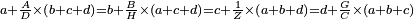
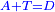
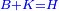

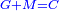

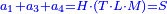



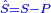
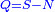

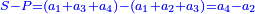
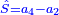
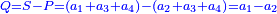

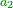 ] is the smallest.
] is the smallest.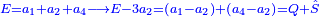
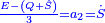

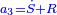
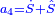

![\scriptstyle{\color{blue}{\left(a_1+a_2+a_3+a_4\right)-\left[a_1+\frac{A}{D}\sdot\left(a_2+a_3+a_4\right)\right]=T\sdot\left(K\times L\times M\right)}}](/mediawiki/images/math/f/2/8/f28561fa101e9defd0856c5899e09d6f.png)
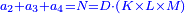
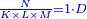
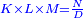
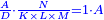
![\scriptstyle{\color{blue}{\frac{\left(a_1+a_2+a_3+a_4\right)-\left[a_1+\frac{A}{D}\sdot\left(a_2+a_3+a_4\right)\right]}{K\times L\times M}=1\sdot T}}](/mediawiki/images/math/d/9/2/d9278fa410fe5bd3bb8780c5a8ac231f.png)
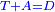
![\scriptstyle{\color{blue}{\left(a_1+a_2+a_3+a_4\right)-\left[a_1+\frac{A}{D}\sdot\left(a_2+a_3+a_4\right)\right]=T\times K\times L\times M}}](/mediawiki/images/math/3/0/0/300f92208b8aea148b938b199fa8aa7f.png)
![\scriptstyle{\color{blue}{\left(a_1+a_2+a_3+a_4\right)-\left[a_2+\frac{B}{H}\sdot\left(a_1+a_3+a_4\right)\right]=K\sdot\left(T\times L\times M\right)=T\times K\times L\times M}}](/mediawiki/images/math/8/a/c/8acc0c02386aa9dad95f7a690dfc3447.png)
![\scriptstyle{\color{blue}{\left(a_1+a_2+a_3+a_4\right)-\left[a_3+\frac{1}{Z}\sdot\left(a_1+a_2+a_4\right)\right]=L\sdot\left(T\times K\times M\right)=T\times K\times L\times M}}](/mediawiki/images/math/0/7/f/07fbc181338f12a6094ede3ec95a6de6.png)
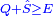
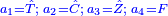
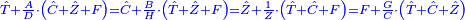
![\scriptstyle{\color{blue}{\begin{align}&\scriptstyle\left(\hat T+\hat C+\hat Z+F\right)-\left[\hat T+\frac{A}{D}\sdot\left(\hat C+\hat Z+F\right)\right]=\left(\hat T+\hat C+\hat Z+F\right)-\left[\hat C+\frac{B}{H}\sdot\left(\hat T+\hat Z+F\right)\right]=\\&\scriptstyle\left(\hat T+\hat C+\hat Z+F\right)-\left[\hat Z+\frac{1}{Z}\sdot\left(\hat T+\hat C+F\right)\right]=\left(\hat T+\hat C+\hat Z+F\right)-\left[F+\frac{G}{C}\sdot\left(\hat T+\hat C+\hat Z\right)\right]=\hat N\end{align}}}](/mediawiki/images/math/6/b/a/6ba8e2bbd3aa1079a45a695a7bb3e6cd.png)

![\scriptstyle{\color{blue}{\left(\hat C+\hat Z+F\right)-\left[\frac{A}{D}\sdot\left(\hat C+\hat Z+F\right)\right]=\hat N=\frac{T}{D}\sdot\left(\hat C+\hat Z+F\right)}}](/mediawiki/images/math/8/4/5/845857841ad6f08d92e0cc776fc3c36e.png)
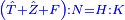
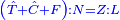
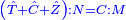
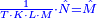
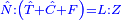
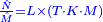
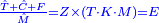

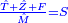
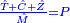
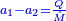
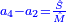
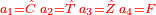
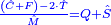
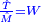
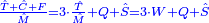
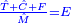
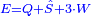
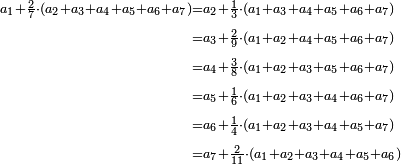
![\scriptstyle{\color{OliveGreen}{7\sdot\left[\left(3-1\right)\sdot\left(9-2\right)\sdot\left(8-3\right)\sdot\left(6-1\right)\sdot\left(4-1\right)\sdot\left(11-2\right)\right]=}}](/mediawiki/images/math/f/1/e/f1e861722b0aca66b8c96a68baefc0ce.png)

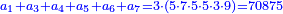
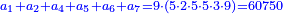

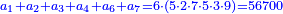



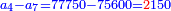

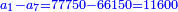

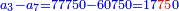
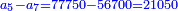

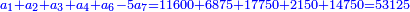

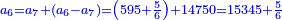

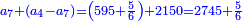
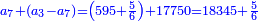

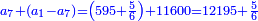

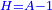


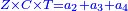



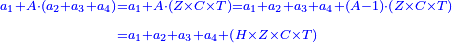
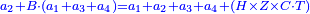

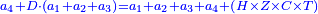

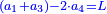




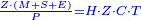
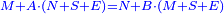
![\scriptstyle{\color{blue}{M+A\sdot\left(N+S+E\right)=\left(M+N+S+E\right)+\left[\left(N+S+E\right)\sdot H\right]}}](/mediawiki/images/math/9/1/a/91af7ac006221f183d4b99feb596fca9.png)
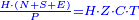
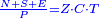
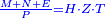
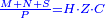

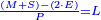
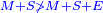
![\scriptstyle\begin{align}\scriptstyle a_1+3\sdot\left(a_2+a_3+a_4+a_5\right)&\scriptstyle=a_2+\left[\left(3+\frac{1}{2}\right)\sdot\left(a_1+a_3+a_4+a_5\right)\right]\\&\scriptstyle=a_3+\left[\left(3+\frac{1}{3}\right)\sdot\left(a_1+a_2+a_4+a_5\right)\right]\\&\scriptstyle=a_4+\left[\left(3+\frac{2}{3}\right)\sdot\left(a_1+a_2+a_3+a_5\right)\right]\\&\scriptstyle=a_5+4\sdot\left(a_1+a_2+a_3+a_4\right)\\\end{align}](/mediawiki/images/math/1/4/1/14188d15cf2276bb455bdf825c1f96a3.png)
![\scriptstyle{\color{OliveGreen}{\left[\left(3+\frac{1}{2}\right)-1\right]\sdot\left[\left(3+\frac{1}{3}\right)-1\right]\sdot\left[\left(3+\frac{2}{3}\right)-1\right]\sdot\left(4-1\right)}}](/mediawiki/images/math/5/a/7/5a7e0f1100e773e16436069c55732c12.png)

![\scriptstyle{\color{OliveGreen}{\left(3-1\right)\sdot\left[\left(3+\frac{1}{3}\right)-1\right]\sdot\left[\left(3+\frac{2}{3}\right)-1\right]\sdot\left(4-1\right)}}](/mediawiki/images/math/b/8/9/b8945f0e33300826a017eb0006fb08ea.png)
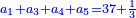
![\scriptstyle{\color{OliveGreen}{\left(3-1\right)\sdot\left[\left(3+\frac{1}{2}\right)-1\right]\sdot\left[\left(3+\frac{2}{3}\right)-1\right]\sdot\left(4-1\right)}}](/mediawiki/images/math/0/3/f/03f4803888a4cb37e8a6ccd796237c2d.png)
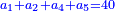
![\scriptstyle{\color{OliveGreen}{\left(3-1\right)\sdot\left[\left(3+\frac{1}{2}\right)-1\right]\sdot\left[\left(3+\frac{1}{3}\right)-1\right]\sdot\left(4-1\right)}}](/mediawiki/images/math/0/f/c/0fc3bb406d02b40c6468749d149c1605.png)

![\scriptstyle{\color{OliveGreen}{\left(3-1\right)\sdot\left[\left(3+\frac{1}{2}\right)-1\right]\sdot\left[\left(3+\frac{1}{3}\right)-1\right]\sdot\left[\left(3+\frac{2}{3}\right)-1\right]}}](/mediawiki/images/math/5/6/4/564d1b9bbb88646ec01da79f98c456df.png)


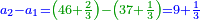

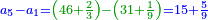
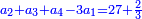
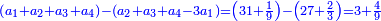
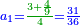
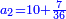
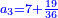
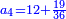
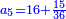
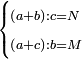
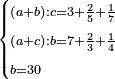
![\scriptstyle{\color{blue}{a_1=\left[\left(3+\frac{2}{5}+\frac{1}{7}\right)\sdot\left(7+\frac{2}{3}+\frac{1}{4}\right)\right]-1=27+\left(\frac{1}{3}\sdot\frac{1}{7}\right)}}](/mediawiki/images/math/2/4/a/24ac89a8bc196c329757a43259b62509.png)
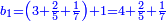
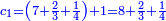

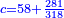
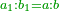
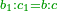

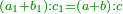

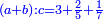

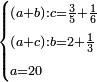
![\scriptstyle{\color{blue}{a_1=\left[\left(\frac{3}{5}+\frac{1}{6}\right)\sdot\left(2+\frac{1}{3}\right)\right]-1=\frac{2}{5}+\frac{1}{3}+\left(\frac{1}{3}\sdot\frac{1}{6}\right)}}](/mediawiki/images/math/c/c/e/cce0fb3dea3b1b8591c7b6a92a28dbec.png)
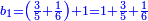
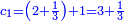
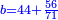
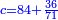
![\scriptstyle{\color{OliveGreen}{\left[\left(a+b\right):c\right]\times\left[\left(a+c\right):b\right]\le1}}](/mediawiki/images/math/b/d/0/bd0234f2658b13af954c8e9fc06aa78e.png)
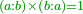
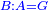
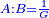

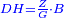
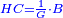

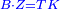

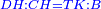
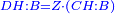
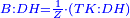

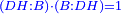
![\scriptstyle{\color{OliveGreen}{\left[\left(a+b\right):c\right]\times\left[\left(a+c\right):b\right]>1}}](/mediawiki/images/math/a/f/0/af0d21745de5318bf13532f9063624e6.png)
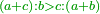
![\scriptstyle{\color{OliveGreen}{\left[\left(a+b\right):c\right]\times\left[c:\left(a+b\right)\right]=1}}](/mediawiki/images/math/e/4/b/e4b29768dbf97f803045f207de566c0e.png)
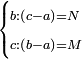
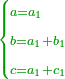
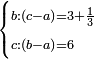
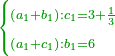
![\scriptstyle{\color{blue}{a_1=\left[\left(3+\frac{1}{3}\right)\sdot6\right]-1=19}}](/mediawiki/images/math/0/3/1/0315920f5be43158ca963f700a9a42e3.png)
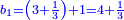
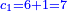
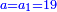


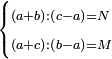
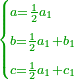

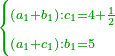
![\scriptstyle{\color{blue}{a_1=\left[\left(4+\frac{1}{2}\right)\sdot5\right]-1=21+\frac{1}{2}}}](/mediawiki/images/math/8/8/a/88a32084717210a422bc31efaed5b615.png)
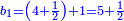


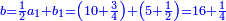

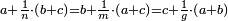
![\scriptstyle{\color{OliveGreen}{\begin{cases}\scriptstyle a_1=g+\left(m-n\right)+\left[\left(n-2\right)\sdot m\sdot g\right]\\\scriptstyle b_1=a_1+\left[2\sdot\left(m-n\right)\sdot\left(g-1\right)\right]\\\scriptstyle c_1=b_1+\left[2\sdot\left(n-1\right)\sdot\left(g-m\right)\right]\end{cases}}}](/mediawiki/images/math/6/1/e/61ec55edce957d940040a72fa46c0418.png)
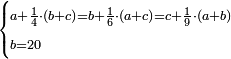
![\scriptstyle{\color{blue}{\begin{cases}\scriptstyle a_1={\color{OliveGreen}{9+\left(6-4\right)+\left[\left(4-2\right)\sdot6\sdot9\right]}}=119\\\scriptstyle b_1={\color{OliveGreen}{119+\left[2\sdot\left(6-4\right)\sdot\left(9-1\right)\right]}}=151\\\scriptstyle c_1={\color{OliveGreen}{151+\left[2\sdot\left(4-1\right)\sdot\left(9-6\right)\right]}}=169\end{cases}}}](/mediawiki/images/math/0/7/4/074a4b1d61e1a0a7570e27584c022daf.png)
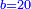

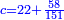
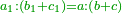
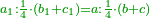
![\scriptstyle{\color{OliveGreen}{\left[a_1+\frac{1}{4}\sdot\left(b_1+c_1\right)\right]:a_1=\left[a+\frac{1}{4}\sdot\left(b+c\right)\right]:a}}](/mediawiki/images/math/3/0/8/308406890c9e763fa74655b23b582e3e.png)
![\scriptstyle{\color{OliveGreen}{\left[b_1+\frac{1}{6}\sdot\left(a_1+c_1\right)\right]:b_1=\left[b+\frac{1}{6}\sdot\left(a+c\right)\right]:b}}](/mediawiki/images/math/1/8/0/1802068c63891b7b5cf28e9ee4ae224b.png)
![\scriptstyle{\color{OliveGreen}{\left[c_1+\frac{1}{9}\sdot\left(a_1+b_1\right)\right]:c_1=\left[c+\frac{1}{9}\sdot\left(a+b\right)\right]:c}}](/mediawiki/images/math/6/5/f/65f8d60060884b91a0d759524433367a.png)
![\scriptstyle{\color{OliveGreen}{\left[a_1+\frac{1}{4}\sdot\left(b_1+c_1\right)\right]:\left[a+\frac{1}{4}\sdot\left(b+c\right)\right]=a_1:a}}](/mediawiki/images/math/4/2/b/42bba98468d5803bcc45ff81098c3594.png)
![\scriptstyle{\color{OliveGreen}{\left[b_1+\frac{1}{6}\sdot\left(a_1+c_1\right)\right]:\left[b+\frac{1}{6}\sdot\left(a+c\right)\right]=b_1:b}}](/mediawiki/images/math/b/b/f/bbf7acabe5a8e23c8a0e41aaf4bd13c7.png)
![\scriptstyle{\color{OliveGreen}{\left[c_1+\frac{1}{9}\sdot\left(a_1+b_1\right)\right]:\left[c+\frac{1}{9}\sdot\left(a+b\right)\right]=c_1:c}}](/mediawiki/images/math/1/1/e/11e9a0acb85fe56fddc0cc15b16bfd04.png)
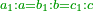
![\scriptstyle{\color{OliveGreen}{\begin{align}\scriptstyle\left[a_1+\frac{1}{4}\sdot\left(b_1+c_1\right)\right]:\left[a+\frac{1}{4}\sdot\left(b+c\right)\right]&\scriptstyle=\left[b_1+\frac{1}{6}\sdot\left(a_1+c_1\right)\right]:\left[b+\frac{1}{6}\sdot\left(a+c\right)\right]\\&\scriptstyle=\left[c_1+\frac{1}{9}\sdot\left(a_1+b_1\right)\right]:\left[c+\frac{1}{9}\sdot\left(a+b\right)\right]\\\end{align}}}](/mediawiki/images/math/0/c/7/0c78b90ed7e7f8da739b75619dac5222.png)
![\scriptstyle{\color{OliveGreen}{\begin{align}&\scriptstyle\left[a_1+\frac{1}{4}\sdot\left(b_1+c_1\right)\right]=\left[b_1+\frac{1}{6}\sdot\left(a_1+c_1\right)\right]=\left[c_1+\frac{1}{9}\sdot\left(a_1+b_1\right)\right]\\&\scriptstyle\longrightarrow\left[a+\frac{1}{4}\sdot\left(b+c\right)\right]=\left[b+\frac{1}{6}\sdot\left(a+c\right)\right]=\left[c+\frac{1}{9}\sdot\left(a+b\right)\right]\\\end{align}}}](/mediawiki/images/math/c/7/3/c73d846798a1afea0ce70d360d60a022.png)