Royslist
From mispar
| Category | Category | Comment | Link | Annotated text |
|---|---|---|---|---|
| algebraic species/additional unknown | דינר | term | חשבון_השטחים#JYwQ | דינר |
| algebraic species/additional unknown | פלס | term | חשבון_השטחים#98Gz | פלס |
| algebra | אלזיברא | term | ספר_ג'יבלי_אלמוקבאלא#NLdc | אלגיברא |
| algebra | אלזיברא | term | ספר_האלזיברא#wsoU | חשבון האלזיברא |
| algebra | אלזיברא | term | ספר_ג'יבלי_אלמוקבאלא#8XWM | אלזיברא |
| algebra | אלג'בר | term | השאלות_החרשות/האלמות#KBDv | אלגבר ואלמוקאבלה |
| algebra | כ.ו.נ./כיוון | term | חשבון_השטחים#1HMQ | חשבונות הכוון |
| algebraic expression/algebraic species | term | חשבון_השטחים#mai1 | מינים | |
| algebraic expression/algebraic species | term | חשבון_השטחים#WRcF | מין | |
| algebra/algebraist | אלג'בר | term | חשבון_השטחים#YnVh | סופרי האלג'בר ואלמקאבלא |
| algebra/algebraist | term | תחבולות_המספר#fi12 | סופרי | |
| algebra/algebraist | ח.כ.מ./חכמי האלג'בר | term | חשבון_השטחים#u2GI | חכמי האלג'בר |
| algebra/algebraist | ח.כ.מ./חכמי האלג'בר | term | תחבולות_המספר#YBWR | חכמי האלגבר |
| algebraic operation/balancing an equation | ש.ו.ה./השואה | term | אגרת_המספר#Mwkf | השואה |
| quartic equation/biquadratic equation | c=ax⁴+√(bx⁴) | ספר_ג'יבלי_אלמוקבאלא#h9il | When numbers are equal to squares of squares and a root of squares of squares:
: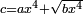 כאשר המספרי' יהיו שוים אל הצינסי מצינסי ואל שרשי צינסי מצינסי
כאשר המספרי' יהיו שוים אל הצינסי מצינסי ואל שרשי צינסי מצינסי | |
| quartic equation/biquadratic equation | 4(x²+8)=x⁴ | תחבולות_המספר#G1Mq | [6] He said: the six problem is as if you are told: we add to a certain square [eight] dirham, then multiply the sum by four dirham and the result is the same as the product of the square [by itself].
: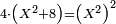 אמ' והשאלה הששית כמו אם יאמרו לך הוספנו על
אמ' והשאלה הששית כמו אם יאמרו לך הוספנו על | |
| quartic equation/biquadratic equation | ax⁴+bx²=c | ספר_ג'יבלי_אלמוקבאלא#Tu7N | When squares of squares plus squares are equal to a number:
: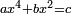 כאשר הצינסי מצינסי וצינסי יהיו שוים אל מספר
כאשר הצינסי מצינסי וצינסי יהיו שוים אל מספר | |
| quartic equation/biquadratic equation | 4(x²+8)=x⁴ | אגרת_המספר#q2Fw | 6) 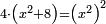 הששית ממון הוספת עליו ח' זוזים והכית המקובץ בארבעה והיה היוצא הכאת הממון בעצמו
הששית ממון הוספת עליו ח' זוזים והכית המקובץ בארבעה והיה היוצא הכאת הממון בעצמו | |
| quartic equation/biquadratic equation | ax⁴=bx²+c | ספר_ג'יבלי_אלמוקבאלא#CLbn | When squares of squares are equal to a number and squares:
: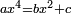 כאשר הצינסי מצינסי יהיו שוים אל המספר והצינסי
כאשר הצינסי מצינסי יהיו שוים אל המספר והצינסי | |
| quartic equation/biquadratic equation | bx²=ax⁴+c | ספר_ג'יבלי_אלמוקבאלא#tkSO | When squares are equal to squares of squares and a root of a number:
: כאשר הצינסי יהיו שוים אל הצינסי מצינסי ואל מספר
כאשר הצינסי יהיו שוים אל הצינסי מצינסי ואל מספר | |
| quartic equation/biquadratic equation | 4(x²+8)=x⁴ | חשבון_השטחים#ZxMx | 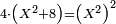 אלגו תוסיף עליו שמנה אדרהם ותכה {{#annot:term|388,1217|vQSL}}המקובץ{{#annotend:vQSL}} על ארבעה אדרהם והיה כמו האלגו על עצמו
אלגו תוסיף עליו שמנה אדרהם ותכה {{#annot:term|388,1217|vQSL}}המקובץ{{#annotend:vQSL}} על ארבעה אדרהם והיה כמו האלגו על עצמו | |
| quadratic equation/canonical equation | term | חשבון_השטחים#hEUg | הששה בקשות | |
| quadratic equation/canonical equation | ח.ל.ק./חלק | term | חשבון_השטחים#rsWG | הששה חלקים |
| canonical equation/compound canonical equation | definition | חשבון_השטחים#FjYV | ושלשה {{#annot: term | #compound canonical equation, #מחובר | y7Pt}}מחוברים{{#annotend:y7Pt}} והם אלגוש ושרשים ישוו מספרים ואלגוש ומספרים ישוו שרשים ושרשים ומספרים ישוו אלגוש | |
| canonical equation/compound canonical equation | ח.ב.ר./מחובר | term | חשבון_השטחים#y7Pt | מחוברים |
| algebraic operation/confrontation | term | חשבון_השטחים#5PgY | קונפרונטאמינטו | |
| algebraic operation/confrontation | ק.ב.ל./הקבלה | definition | אגרת_המספר#pj9j | the reduction is to subtract each species from its similar until there are no two types of the same species on both sides [of the equation] אמ' וההקבלה היא לגרוע כל מין מהדומה לו עד שלא יהיה בצדדין שני מינים מסוג אחד |
| algebraic operation/confrontation | ק.ב.ל./הקבלה | term | אגרת_המספר#eRhw | הקבלה |
| algebraic operation/confrontation | term | תחבולות_המספר#ZrCF | איקונפרונטאמיינטו | |
| algebraic operation/confrontation | כ.ו.נ./כיוון | term | תחבולות_המספר#O1Uz | כוון |
| algebraic operation/confrontation | term | חשבון_השטחים#FhaB | קונפרונטאמיינטו | |
| equation/cubic equation | ax³=³√c | ספר_ג'יבלי_אלמוקבאלא#eOI5 | It is when cubes are equal to a cube root of the numbers:
:![\scriptstyle ax^3=\sqrt[3]{c}](/mediawiki/images/math/a/6/4/a64e6eaeaf2d5f64610f741998d43d86.png) וזהו כאשר המעוקבי' יהיו שוים אל שרש מעו' ממספרי'
וזהו כאשר המעוקבי' יהיו שוים אל שרש מעו' ממספרי' | |
| algebraic expression/equation | term | ספר_ג'יבלי_אלמוקבאלא#zCuO | שאלות | |
| algebraic expression/equation | term | ספר_ג'יבלי_אלמוקבאלא#gmyo | שאלה | |
| algebraic species/higher power of x | term | חשבון_השטחים#YTVZ | אלגו אלגו אלגו אלגו | |
| algebraic species/higher power of x | term | ספר_ג'יבלי_אלמוקבאלא#UeHi | צינסי דצינסי | |
| algebraic species/higher power of x | term | ספר_ג'יבלי_אלמוקבאלא#rRuk | צינסי מצינסי | |
| algebraic species/higher power of x | term | ספר_ג'יבלי_אלמוקבאלא#iVFX | צינסו מצינסו | |
| algebraic species/higher power of x | term | חשבון_השטחים#HyOd | האלגו מהאלגו | |
| algebraic species/higher power of x | term | ספר_האלזיברא#IRdl | מרובע המרובע | |
| algebraic species/higher power of x | term | ספר_ג'יבלי_אלמוקבאלא#9Mat | צינסו דצינסו | |
| algebraic species/higher power of x | term | ספר_ג'יבלי_אלמוקבאלא#Ae4R | מעו' המעו' | |
| algebraic species/higher power of x | term | ספר_ג'יבלי_אלמוקבאלא#R4d1 | צינסי דצינסי דצינסי | |
| algebraic species/higher power of x | term | ספר_ג'יבלי_אלמוקבאלא#ioCC | צינסו דצינסו מצינסו | |
| algebraic species/higher power of x | term | חשבון_השטחים#f1b2 | אלגוש מאלגו | |
| algebraic species/higher power of x | term | חשבון_השטחים#Rpq0 | אלגוש אלגו | |
| algebraic species/higher power of x | term | חשבון_השטחים#7JWO | קוביקא מקוביקא | |
| algebraic species/higher power of x | term | ספר_ג'יבלי_אלמוקבאלא#nLSh | מעו' ממעו' | |
| algebraic species/higher power of x | term | ספר_ג'יבלי_אלמוקבאלא#hdFg | צינסו מצינסו מצינסו | |
| algebraic species/higher power of x | term | ספר_ג'יבלי_אלמוקבאלא#yHS1 | צינסו מצינסו מצינסו מצינסו | |
| algebraic species/higher power of x | term | ספר_ג'יבלי_אלמוקבאלא#RcAh | צינסי מצינסי מצינסי מצינסי | |
| algebraic species/higher power of x | term | חשבון_השטחים#oORe | מעוקבים ממעוקב | |
| algebraic species/higher power of x | term | חשבון_השטחים#E8pq | מעוקב ממעוקב | |
| algebraic species/higher power of x | term | חשבון_השטחים#z61g | אלגו אלגו מוכה בדבר | |
| algebraic species/higher power of x | term | ספר_ג'יבלי_אלמוקבאלא#IHxD | צינסי מצינסי מצינסי | |
| algebraic species/higher power of x | term | ספר_ג'יבלי_אלמוקבאלא#3zl2 | מעוקבי' ממעוקבי' | |
| algebraic species/higher power of x | term | ספר_האלזיברא#RTpD | מעקב המעקב | |
| algebraic species/higher power of x | term | ספר_האלזיברא#IpzF | קוּבוּ דֵקוּבוּ | |
| algebraic species/higher power of x | term | ספר_האלזיברא#nlXb | צֵינְסו דֵצֵינְסו | |
| equation/indeterminate equation | 2x+49-x²=n² | השאלות_החרשות/האלמות#bkYa | 21) If you are told: a square, if you subtract it from its two roots plus 49 dirham, the remainder has a root.
: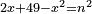 כא ואם יאמרו לך אלגו אם תגרעהו משני שרשיו ומ"ט דרהמי יחזיק הנשאר שרש
כא ואם יאמרו לך אלגו אם תגרעהו משני שרשיו ומ"ט דרהמי יחזיק הנשאר שרש | |
| equation/indeterminate equation | x²+(2-x)=n²,x²-(3-x)=m² | השאלות_החרשות/האלמות#xP6r | 38) If you are told: a square that has a root, if you add two dirham minus the root of the square to it, it has a root; and if you subtract three minus the root of the square from it, it has a root.
: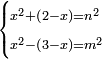 לח ואם יאמרו לך אלגו המחזיק שרש אם תוסיף עליו שני דרהמי פחות שרש האלגו יחזיק שרש
לח ואם יאמרו לך אלגו המחזיק שרש אם תוסיף עליו שני דרהמי פחות שרש האלגו יחזיק שרשואם תגרע ממנו שלשה פחות שרש האלגו יחזיק שרש | |
| equation/indeterminate equation | x²-(x-1)=n²,x²-(1-x)=m² | השאלות_החרשות/האלמות#Wdk5 | 37) If you are told: a square that has a root, if you subtract one dirham minus the root of the square from it, it has a root; [and if you subtract its root minus one dirham from it, it has a root].
: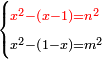 לז ואם יאמרו לך אלגו המחזיק שרש אם תגרע ממנו דרהם אחד פחות שרש האלגו יחזיק הנשאר שרש
לז ואם יאמרו לך אלגו המחזיק שרש אם תגרע ממנו דרהם אחד פחות שרש האלגו יחזיק הנשאר שרש | |
| equation/indeterminate equation | x²+3x+1=n²,x²-(3x-2)=m² | השאלות_החרשות/האלמות#43ha | 36) If you are told: a square that has a root, if you add to it its three roots and one dirham, it has a root; and if you subtract its three roots minus two dirham from it, the remainder has a root.
: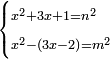 לו ואם יאמרו לך אלגו שיחזיק שרש אם תוסיף עליו שלשת שרשיו ודרהם אחד יחזיק שרש
לו ואם יאמרו לך אלגו שיחזיק שרש אם תוסיף עליו שלשת שרשיו ודרהם אחד יחזיק שרשואם תגרע ממנו שלשת שרשיו פחות שני דרהמי יחזיק מה שישאר שרש | |
| equation/indeterminate equation | x²-2x=n²,x²-2x+√(x²-2x)=m² | השאלות_החרשות/האלמות#65Ct | 35) If you are told: a square that has a root, if you subtract its two roots from it, it has a root; and if you add to the remainder its root, it has a root.
: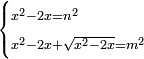 לה ואם יאמרו לך אלגו המחזיק שרש אם תגרע ממנו שני שרשיו יחזיק שרש
לה ואם יאמרו לך אלגו המחזיק שרש אם תגרע ממנו שני שרשיו יחזיק שרשואם תוסיף על הנשאר שרשו יחזיק שרש | |
| equation/indeterminate equation | x²+4x=n²,x²-(2x+1)=m² | השאלות_החרשות/האלמות#1vZf | 34) If you are told: a square that has a root, if you add its four roots to it, it has a root; and if you subtract its two roots plus one dirham from it, the remainder has a root.
: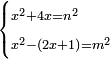 לד ואם יאמרו לך אלגו שיחזיק שרש אם תוסיף עליו ארבעת שרשיו יחזיק שרש
לד ואם יאמרו לך אלגו שיחזיק שרש אם תוסיף עליו ארבעת שרשיו יחזיק שרשואם תגרע ממנו שני שרשיו ודרהם אחד יחזיק הנשאר שרש | |
| equation/indeterminate equation | x²-5=n²,x²-5+√(x²-5)=m² | השאלות_החרשות/האלמות#6x8t | 33) If you are told: a square that has a root, if you subtract five dirham from it, the remainder has a root; and if you add to the remainder its root, the sum has a root.
: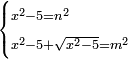 לג ואם יאמרו לך אלגו המחזיק שרש אם תגרע ממנו חמשה דרהמי יחזיק הנשאר שרש
לג ואם יאמרו לך אלגו המחזיק שרש אם תגרע ממנו חמשה דרהמי יחזיק הנשאר שרשואם תוסיף על הנשאר שרשו יחזיק המקובץ שרש | |
| equation/indeterminate equation | x²+x=n²,x²+1=m² | השאלות_החרשות/האלמות#eNRx | 32) If you are told: a square that has a root, if you add its root to it, it has a root; and if you add to one dirham to it, it has a root.
: לב ואם יאמרו לך אלגו המחזיק שרש אם תוסיף עליו שרשו יחזיק שרשו
לב ואם יאמרו לך אלגו המחזיק שרש אם תוסיף עליו שרשו יחזיק שרשוואם תוסיף עליו דרהם אחד יחזיק שרש | |
| equation/indeterminate equation | x²-4x=n²,x²-4x-2√(x²-4x)=m² | השאלות_החרשות/האלמות#vAyv | 31) If you are told: a square, if you subtract its four roots from it, it has a root; and if you subtract from the remainder its two roots, it has a root.
: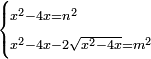 לא ואם יאמרו לך אלגו אם
לא ואם יאמרו לך אלגו אם ואם תגרע ואם תגרע מהנשאר שני שרשיו יחזיק שרש | |
| equation/indeterminate equation | x²+4x=n²,x²+4x+2√(x²+4x)=m² | השאלות_החרשות/האלמות#2scj | 30) If you are told: a square that has a root, if you add its four roots to it, it has a root; and if you add to the sum its two roots, it has a root.
: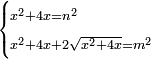 ל וכן אם יאמרו לך אלגו המחזיק שרש
ל וכן אם יאמרו לך אלגו המחזיק שרשאם תוסיף עליו ארבעת שרשיו יחזיק שרש ואם תוסיף על המתקבץ שני שרשיו יחזיק שרש | |
| equation/indeterminate equation | x²+2x=n²,x²+2x+√(x²+2x)=m² | השאלות_החרשות/האלמות#Csxg | 29) If you are told: a square that has a root, if you add two roots to it, it has a root; and if you add to the sum its root, it has a root.
: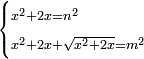 כט כאשר יאמרו לך אלגו המחזיק שרש אם תוסיף עליו שני שרשים יחזיק שרש
כט כאשר יאמרו לך אלגו המחזיק שרש אם תוסיף עליו שני שרשים יחזיק שרשואם תוסיף על המקובץ שרשו יחזיק שרש | |
| equation/indeterminate equation | x²+3x=n²,x²+3x+6√(x²+3x)=m² | השאלות_החרשות/האלמות#RKqA | 28) If you are told: a square that has a root, if you add its three roots to it, it has a root; and if you add to the sum its six roots, it has a root.
: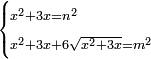 כח וכן אם יאמרו לך אלגו המחזיק שרש אם תוסיף עליו שלשת שרשיו יחזיק שרש
כח וכן אם יאמרו לך אלגו המחזיק שרש אם תוסיף עליו שלשת שרשיו יחזיק שרשואם תקבץ עם העולה ששת שרשיו יחזיק שרש | |
| equation/indeterminate equation | x²+2x=n²,x²+2x+3√(x²+2x)=m² | השאלות_החרשות/האלמות#Xpj1 | 27) If you are told: a square that has a root, if you add its two roots to it, it has a root; and if you add to the sum its three roots, it has a root.
: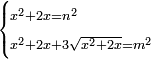 כז ואם יאמרו לך אלגו המחזיק שרש אם תוסיף עליו שני שרשיו יחזיק שרש
כז ואם יאמרו לך אלגו המחזיק שרש אם תוסיף עליו שני שרשיו יחזיק שרשואם תוסיף על המקובץ שלשת שרשיו יחזיק שרש | |
| equation/indeterminate equation | 260-6x-x²=n² | השאלות_החרשות/האלמות#63F7 | 26) If you are told: a square that has a root, if you subtract it from 260 minus six roots, the remainder has a root.
: כו ואם יאמרו לך אלגו המחזיק שרש אם תגרעהו מר"ס פחות ששה שרשים יחזיק הנשאר שרש
כו ואם יאמרו לך אלגו המחזיק שרש אם תגרעהו מר"ס פחות ששה שרשים יחזיק הנשאר שרש | |
| equation/indeterminate equation | x²+1=10x-8 | השאלות_החרשות/האלמות#g7c7 | 25) If you are told: a square and one dirham equal ten roots minus eight dirham.
: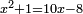 כה ואם יאמרו לך אלגו ודרהם אחד ישוו עשרה שרשים פחות שמנה דרהמי
כה ואם יאמרו לך אלגו ודרהם אחד ישוו עשרה שרשים פחות שמנה דרהמי | |
| equation/indeterminate equation | 10x-8-x²=n² | השאלות_החרשות/האלמות#JrVS | 24) If you are told: a square that has a root, if you subtract it from its ten roots minus eight dirham, the remainder has a root.
: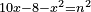 כד ואם יאמרו לך אלגו שיחזיק שרש אם תגרעהו מעשרת שרשיו פחות שמנה
כד ואם יאמרו לך אלגו שיחזיק שרש אם תגרעהו מעשרת שרשיו פחות שמנה | |
| equation/indeterminate equation | x²+2x=n²,x²-3x=m² | השאלות_החרשות/האלמות#ltjx | 23) If you are told: a square, if you add its two roots to it, it has a root; and if you subtract its three roots from it, it has a root.
: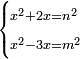 כג ואם יאמרו לך אלגו אם תוסיף עליו שני שרשיו יחזיק שרש ואם תגרע ממנו שלשה שרשיו יחזיק שרש
כג ואם יאמרו לך אלגו אם תוסיף עליו שני שרשיו יחזיק שרש ואם תגרע ממנו שלשה שרשיו יחזיק שרש | |
| equation/indeterminate equation | x²+x=n²,x²-x=m² | השאלות_החרשות/האלמות#MUv8 | 22) If you are told: a square, if you add its root to it, the sum has a root; and if you subtract its root from it, the remainder has a root.
: כב ואם יאמרו לך אלגו אם תוסיף עליו שרשו יהיה למתקבץ שרש ואם תגרע ממנו שרשו יחזיק הנשאר שרש
כב ואם יאמרו לך אלגו אם תוסיף עליו שרשו יהיה למתקבץ שרש ואם תגרע ממנו שרשו יחזיק הנשאר שרש | |
| equation/indeterminate equation | x²+x+1=n²,x²+2x+2=m² | השאלות_החרשות/האלמות#YEF9 | 39) If you are told: a square that has a root, if you add its root plus one dirham to it, it has a root; and if you add to the square its two roots plus two dirham, it has a root.
: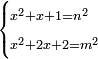 לט ואם יאמרו לך אלגו המחזיק שרש אם תוסיף עליו שרשו ודרהם אחד יחזיק שרש
לט ואם יאמרו לך אלגו המחזיק שרש אם תוסיף עליו שרשו ודרהם אחד יחזיק שרשואם תוסיף על האלגו שני שרשיו ושני דרהמי יחזיק שרש | |
| equation/indeterminate equation | 8x+x²=n²,2x-x²=m² | השאלות_החרשות/האלמות#62Ll | 20) If you are told: a square, if you add its eight roots to it, it has a root; and if you subtract it from its two roots, the remainder has a root.
: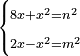 כ ואם יאמרו לך אלגו אם תוסיף עליו שמנה שרשיו יחזיק שרש ואם תגרעהו משני שרשיו יהיה לנשאר שרש
כ ואם יאמרו לך אלגו אם תוסיף עליו שמנה שרשיו יחזיק שרש ואם תגרעהו משני שרשיו יהיה לנשאר שרש | |
| equation/indeterminate equation | 8x+109-x²=n² | השאלות_החרשות/האלמות#tMRx | 19) If you are told: a square, if you subtract it from its eight roots plus 109 dirham, it has a root.
: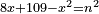 יט ואם יאמרו לך אלגו אם תגרעהו משמנה שרשים וק"ט דרהמי יחזיק שרש
יט ואם יאמרו לך אלגו אם תגרעהו משמנה שרשים וק"ט דרהמי יחזיק שרש | |
| equation/indeterminate equation | 10+x²=n²,10-x²=m² | השאלות_החרשות/האלמות#rWQ1 | 18) If you are told: a square, if you add it to ten, it has a root; and if you subtract it from ten, it has a root.
: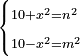 יח ואם יאמרו לך אלגו אם תוסיפהו על עשרה יחזיק שרש ואם תגרעהו 70vמעשרה יחזיק שרש
יח ואם יאמרו לך אלגו אם תוסיפהו על עשרה יחזיק שרש ואם תגרעהו 70vמעשרה יחזיק שרש | |
| equation/indeterminate equation | 20+x²=n²,30+x²=m² | השאלות_החרשות/האלמות#czE5 | 17) If you are told: a square, if you add it to twenty, it has a root; and if you add it to thirty, it has a root.
: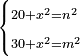 יז ואם יאמרו לך אלגו אם תוסיפהו על עשרים יחזיק שרש ואם תוסיפיהו על שלשים יחזיק שרש
יז ואם יאמרו לך אלגו אם תוסיפהו על עשרים יחזיק שרש ואם תוסיפיהו על שלשים יחזיק שרש | |
| equation/indeterminate equation | 10-x²=n²,20-x²=m² | השאלות_החרשות/האלמות#EaVV | 16) If you are told: a square, if you subtract it from ten dirham, the remainder has a root; and if you subtract it from twenty, the remainder has a root.
: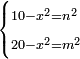 יו ואם יאמרו לך אלגו אם תגרעהו מעשרה דרהמי יחזיק הנשאר שרש ואם תגרעהו מעשרים יחזיק הנשאר שרש
יו ואם יאמרו לך אלגו אם תגרעהו מעשרה דרהמי יחזיק הנשאר שרש ואם תגרעהו מעשרים יחזיק הנשאר שרש | |
| equation/indeterminate equation | 3-x²=n²,2+x²=m² | השאלות_החרשות/האלמות#6Zd3 | 15) If you are told: a square, if you subtract it from three dirham, the remainder has a root; and if you add it to two dirham, the sum has a root.
: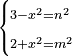 טו ואם יאמרו לך מרובע אם תגרעהו משלשה דרהמי יחזיק הנשאר שרש ואם תוסיפהו על שני דרהמי יהיה למתקבץ שרש
טו ואם יאמרו לך מרובע אם תגרעהו משלשה דרהמי יחזיק הנשאר שרש ואם תוסיפהו על שני דרהמי יהיה למתקבץ שרש | |
| equation/indeterminate equation | x²+y²=n² | השאלות_החרשות/האלמות#Onda | 12) If you are told: divide five dirham into two parts, so that each part has a root.
: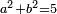 יב אם יאמרו לך חמשה דרהמי תחלקם לשני חלקים ולכל חלק שרש
יב אם יאמרו לך חמשה דרהמי תחלקם לשני חלקים ולכל חלק שרש | |
| equation/indeterminate equation | x+x²=n²,x-x²=m² | השאלות_החרשות/האלמות#Fofb | 11) If you are told: a square that has a root, if you add its root to it, it has a root; and if you subtract the square from its root, the remainder has a root.
: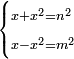 יא ואם יאמרו לך מרובע המחזיק שרש אם תוסיף עליו שרשו יחזיק שרש ואם תגרע המרובע משרשו יהיה לנשאר שרש
יא ואם יאמרו לך מרובע המחזיק שרש אם תוסיף עליו שרשו יחזיק שרש ואם תגרע המרובע משרשו יהיה לנשאר שרש | |
| equation/indeterminate equation | x-x²=n² | השאלות_החרשות/האלמות#hdU1 | 10) If you are told: a square that has a root, if you subtract it from its root, the remainder has a root.
: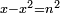 67vי ואם יאמרו לך מרובע המחזיק שרש אם תגרעהו מן שרשו יהיה לנשאר שרש
67vי ואם יאמרו לך מרובע המחזיק שרש אם תגרעהו מן שרשו יהיה לנשאר שרש | |
| equation/indeterminate equation | x²-y=n²,x²-1½y=m² | השאלות_החרשות/האלמות#CUHD | When you wish to find a number that has a root, such that when you subtract from it another number, the remainder has a root; and if you subtract from it again the other number and its half, the remainder has a root.
: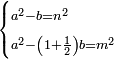 וכאשר תרצה למצוא מספר המחזיק שרש שכאשר תגרע ממנו מספר אחר יהיה לנשאר שרש ואם תגרע עוד ממנו כמו המספר האחר וכמו חציו יהיה לנשאר שרש
וכאשר תרצה למצוא מספר המחזיק שרש שכאשר תגרע ממנו מספר אחר יהיה לנשאר שרש ואם תגרע עוד ממנו כמו המספר האחר וכמו חציו יהיה לנשאר שרש | |
| equation/indeterminate equation | x²-2x=n²,x²-3x=m² | השאלות_החרשות/האלמות#65Jm | 9) If you are told: a square that has a root, if you subtract its two roots from it, the remainder has a root; and if you subtract its three roots from the square, the remainder has a root.
: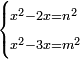 ט וכן אם יאמרו לך מרובע המחזיק שרש אם תגרע ממנו שני שרשים יחזיק הנשאר שרש ואם תגרע מהמרובע שלשה שרשים יחזיק הנשאר שרש
ט וכן אם יאמרו לך מרובע המחזיק שרש אם תגרע ממנו שני שרשים יחזיק הנשאר שרש ואם תגרע מהמרובע שלשה שרשים יחזיק הנשאר שרש | |
| equation/indeterminate equation | 49+x=n²,49+2x=m² | השאלות_החרשות/האלמות#Y9EL | If you wish to know the number that when you add it to 49, it has a root; and if you add it to it twice, it has a root.
: ואם רצית לדעת המספר שכאשר תוסיפהו על מ"ט יחזיק שרש ואם תוסיפהו עליו שני פעמים יחזיק שרש
ואם רצית לדעת המספר שכאשר תוסיפהו על מ"ט יחזיק שרש ואם תוסיפהו עליו שני פעמים יחזיק שרש | |
| equation/indeterminate equation | x²+x=n²,x²+3x=m² | השאלות_החרשות/האלמות#5iEI | 8) If you are told: a square, if you add its root to it, it has a root; and if you add its three roots to it, it has a root.
: ח וכן אם יאמרו לך אלגו אם תוסיף עליו שרשו יחזיק שרש ואם תוסיף עליו שלשה שרשיו יחזיק שרש
ח וכן אם יאמרו לך אלגו אם תוסיף עליו שרשו יחזיק שרש ואם תוסיף עליו שלשה שרשיו יחזיק שרש | |
| equation/indeterminate equation | x²+x=n²,x²+2x=m² | השאלות_החרשות/האלמות#9AQM | 7) If you are told: a square that has a root, if you add its root to it, it has a root; and if you add its two roots to it, it has a root.
: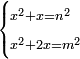 ז ואם יאמרו לך אלגו שיחזיק שרש אם תוסיף עליו שרשו יחזיק שרש ואם תוסיף עליו אלגו שני שרשיו יחזיק שרש
ז ואם יאמרו לך אלגו שיחזיק שרש אם תוסיף עליו שרשו יחזיק שרש ואם תוסיף עליו אלגו שני שרשיו יחזיק שרש | |
| equation/indeterminate equation | x²-8x-30=n² | השאלות_החרשות/האלמות#67aP | 6) If you are told: a square that has a root, if you subtract from it eight times its root and thirty dirham, the remainder has a root.
: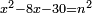 ו ואם יאמרו לך אלגו המחזיק שרש אם תגרע ממנו שרשו שמנה פעמי' ושלשים דרהמי יחזיק מה שישאר שרש
ו ואם יאמרו לך אלגו המחזיק שרש אם תגרע ממנו שרשו שמנה פעמי' ושלשים דרהמי יחזיק מה שישאר שרש | |
| equation/indeterminate equation | x²-10=n² | השאלות_החרשות/האלמות#mV1w | 2) When you are told: a square that has a root, if you subtract ten dirham from it, what remains has a root.
: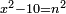 ב וכאשר יאמרו לך אלגו המחזיק שרש אם תגרע ממנו עשרה דרהמי יחזיק מה שישאר שרש
ב וכאשר יאמרו לך אלגו המחזיק שרש אם תגרע ממנו עשרה דרהמי יחזיק מה שישאר שרש | |
| equation/indeterminate equation | x²+10x+20=n² | השאלות_החרשות/האלמות#x5bu | 5) If you are told: a square that has a root, if you add to it ten times its root plus ten dirham, it has a root.
: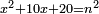 ה ואם יאמרו לך אלגו המחזיק שרש אם תוסיף עליו עשרה פעמים שרשו ועשרה דרהמי יחזיק שרש
ה ואם יאמרו לך אלגו המחזיק שרש אם תוסיף עליו עשרה פעמים שרשו ועשרה דרהמי יחזיק שרש | |
| equation/indeterminate equation | x²-6x=n² | השאלות_החרשות/האלמות#LfMV | 4) If you are told: a square that has a root, when we subtract six times its root from it, it has a root.
: ד ואם יאמרו לך אלגו מחזיק שרש כאשר גרענו ממנו שרשו ששה פעמים יחזיק שרש
ד ואם יאמרו לך אלגו מחזיק שרש כאשר גרענו ממנו שרשו ששה פעמים יחזיק שרש | |
| equation/indeterminate equation | x²+3x=n² | השאלות_החרשות/האלמות#L1j9 | 3) If you are told: a square that has a root, if you add three times its root to it, it has a root. How much is the square?
: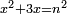 ג ואם יאמרו לך אלגו המחזיק שרש אם תוסיף עליו שרשו שלשה פעמים יחזיק שרש כמה יהיה האלגו
ג ואם יאמרו לך אלגו המחזיק שרש אם תוסיף עליו שרשו שלשה פעמים יחזיק שרש כמה יהיה האלגו | |
| equation/indeterminate equation | x²+5=n² | השאלות_החרשות/האלמות#rm8m | 1) When you are told: a square that has a root, if you add five to it, it has a root. How much is the square?
: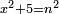 א כאשר יאמרו לך אלגו שמחזיק שרש אם תוסיף עליו חמשה יחזיק שרש כמה הוא האלגו
א כאשר יאמרו לך אלגו שמחזיק שרש אם תוסיף עליו חמשה יחזיק שרש כמה הוא האלגו | |
| equation/linear equation | bx=³√c | ספר_ג'יבלי_אלמוקבאלא#NHZd | When things are equal to a cube root of the numbers:
:![\scriptstyle bx=\sqrt[3]{c}](/mediawiki/images/math/4/e/c/4eced3ba1c8f51bf47cf911659b6e201.png) כאשר הדברי' יהיו שוי' אל שרש מעוק' ממספרי'
כאשר הדברי' יהיו שוי' אל שרש מעוק' ממספרי' | |
| equation/linear equation | c=³√bx | ספר_ג'יבלי_אלמוקבאלא#jGFm | When numbers are equal to a cube root of a thing:
:![\scriptstyle c=\sqrt[3]{bx}](/mediawiki/images/math/5/b/4/5b40a2184fb3c6324ff5fe2eedb9da0b.png) כאשר המספרי' יהיו שוים אל שרש מעו' מדבר
כאשר המספרי' יהיו שוים אל שרש מעו' מדבר | |
| algebraic operation/multiplication of algebraic expressions | 3x×6 | תחבולות_המספר#NmR4 | If you are told: how much is the result of multiplying three things by six dirham?
: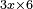 אמ' ואם יאמרו לך כמה יעלה מהכאת שלשה דברי' בששה דרהמי
אמ' ואם יאמרו לך כמה יעלה מהכאת שלשה דברי' בששה דרהמי | |
| algebraic operation/multiplication of algebraic expressions | (10+x)×(10+x) | תחבולות_המספר#e5Fz | If you are told: how much is the product of ten dirham plus a thing by ten dirham plus a thing?
: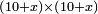 אמר ואם יאמרו לך כמה יעלה מהכאת עשרה דרהמי ודבר בעשרה דרהמי ודבר
אמר ואם יאמרו לך כמה יעלה מהכאת עשרה דרהמי ודבר בעשרה דרהמי ודבר | |
| algebraic operation/multiplication of algebraic expressions | (10-x)×(10-x) | תחבולות_המספר#wU5C | If you are told: how much is the product of ten dirham minus a thing by ten dirham minus a thing?
: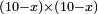 אמ' ואם יאמרו לך כמה יהיה מהכאת עשרה אדרהמי פחות דבר בעשרה אדרהמי פחות דבר
אמ' ואם יאמרו לך כמה יהיה מהכאת עשרה אדרהמי פחות דבר בעשרה אדרהמי פחות דבר | |
| algebraic operation/multiplication of algebraic expressions | (10+⅔x)×(3-6x) | חשבון_השטחים#EjPJ | 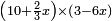 ואם יאמרו לך כמה יהיו עשרה אדרהמיש ושני שלישי דבר על שלשה אדרהמיש פחות ששה דברים
ואם יאמרו לך כמה יהיו עשרה אדרהמיש ושני שלישי דבר על שלשה אדרהמיש פחות ששה דברים | |
| algebraic operation/multiplication of algebraic expressions | (10+x)×(x-10) | חשבון_השטחים#xrWT |  ואם יאמרו לך כמה יהיה עשרה אדרהמיש ודבר על דבר פחות עשרה אדרהמיש
ואם יאמרו לך כמה יהיה עשרה אדרהמיש ודבר על דבר פחות עשרה אדרהמיש | |
| algebraic operation/multiplication of algebraic expressions | (10+x)×(10-x) | חשבון_השטחים#9LHU | 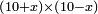 ואם יאמרו לך עשרה אדרהמיש ודבר על עשרה אדרהמיש פחות דבר
ואם יאמרו לך עשרה אדרהמיש ודבר על עשרה אדרהמיש פחות דבר | |
| algebraic operation/multiplication of algebraic expressions | (10-x)×(10-x) | חשבון_השטחים#w9JD | 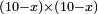 ואם יאמרו לך כמה יהיה עשרה אדרהמיש פחות דבר בעשרה אדרהמיש פחות דבר
ואם יאמרו לך כמה יהיה עשרה אדרהמיש פחות דבר בעשרה אדרהמיש פחות דבר | |
| algebraic operation/multiplication of algebraic expressions | (10+x)×(10+x) | חשבון_השטחים#aUXi | 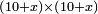 ואם יאמרו לך כמה יהיו עשרה אדרהמיש ודבר בעשרה אדרהמיש ודבר
ואם יאמרו לך כמה יהיו עשרה אדרהמיש ודבר בעשרה אדרהמיש ודבר | |
| algebraic operation/multiplication of algebraic expressions | (10-x)×x | חשבון_השטחים#TINt | 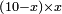 ואם יאמרו לך עשרה אדרהמיש פחות דבר אחד על דבר אחד
ואם יאמרו לך עשרה אדרהמיש פחות דבר אחד על דבר אחד | |
| algebraic operation/multiplication of algebraic expressions | 3x×6 | חשבון_השטחים#6cOe | 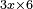 ואם יאמרו כמה יהיו שלשה דברים בששה אדרהמיש
ואם יאמרו כמה יהיו שלשה דברים בששה אדרהמיש | |
| algebraic operation/multiplication of algebraic expressions | (10+x)×x | חשבון_השטחים#mpes | 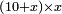 ואם יאמרו לך כמה יהיה עשרה אהדרמיש ודבר בדבר אחד
ואם יאמרו לך כמה יהיה עשרה אהדרמיש ודבר בדבר אחד | |
| algebraic operation/multiplication of algebraic expressions | 2x×2x | חשבון_השטחים#mF0Q | 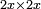 ואשים ענין זה בהכאת שני דברים בשני דברים
ואשים ענין זה בהכאת שני דברים בשני דברים | |
| algebraic operation/multiplication of algebraic expressions | (10+x)×(10-x) | תחבולות_המספר#FNrC | If you are told: how much is the product of ten dirham plus a thing by ten dirham minus a thing?
: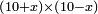 אמ' ואם יאמרו לך כמה יעלה מהכאת עשרה דרהמי ודבר בעשרה דרהמי פחות דבר
אמ' ואם יאמרו לך כמה יעלה מהכאת עשרה דרהמי ודבר בעשרה דרהמי פחות דבר | |
| algebraic operation/multiplication of algebraic expressions | (10+x)×x | תחבולות_המספר#Dvm5 | If you are told: how much is the product of ten dirham plus a thing by one thing?
: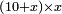 אמ' ואם יאמרו לך כמה יהיה מהכאת עשרה דרהמי ודבר בדבר אחד
אמ' ואם יאמרו לך כמה יהיה מהכאת עשרה דרהמי ודבר בדבר אחד | |
| algebraic operation/multiplication of algebraic expressions | (10+⅔x)×(3-6x) | תחבולות_המספר#VDLn | He said: if you are told: how much is the product of ten dirham and two-thirds of a thing by three dirham minus six things?
: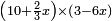 אמר ואם יאמרו לך כמה יהיה מהכאת עשרה דרהמי ושני שלישי דבר על שלשה דרהמי פחות ששה דברים
אמר ואם יאמרו לך כמה יהיה מהכאת עשרה דרהמי ושני שלישי דבר על שלשה דרהמי פחות ששה דברים | |
| algebraic operation/multiplication of algebraic expressions | (10-x)×x | תחבולות_המספר#EDhK | If you are told: how much is the product of ten dirham minus a thing by a thing?
: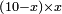 אמ' ואם יאמרו לך כמה יעלה מהכאת עשרה דרהמי {{#annot:term|879,1366|9fZc}}פחות{{#annotend:9fZc}} דבר אחד בדבר אחד
אמ' ואם יאמרו לך כמה יעלה מהכאת עשרה דרהמי {{#annot:term|879,1366|9fZc}}פחות{{#annotend:9fZc}} דבר אחד בדבר אחד | |
| algebraic operation/multiplication of algebraic expressions | 2x×2x | תחבולות_המספר#AfDN | 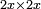 ונעמיד זה הענין בהכאת שני דברי' בשני דברים
ונעמיד זה הענין בהכאת שני דברי' בשני דברים | |
| algebraic operation/multiplication of algebraic expressions | (10+x)×(x-10) | תחבולות_המספר#Ga64 | If you are told: how much is the product of ten dirham plus a thing by a thing minus ten dirham?
: אמ' ואם יאמרו לך כמה העולה מהכאת עשרה דרהמי ודבר בדבר פחות עשרה דרהמי
אמ' ואם יאמרו לך כמה העולה מהכאת עשרה דרהמי ודבר בדבר פחות עשרה דרהמי | |
| algebraic operation/normalization | ש.ל.מ./השלמה | term | חשבון_השטחים#79UI | השלמתך הוא עד שיהיו אלגו שלם |
| algebraic species/number (algebraic context) | דרהם | term | חשבון_השטחים#qDuw | אדרהם |
| algebraic species/number (algebraic context) | ס.פ.ר./מספר | term | תחבולות_המספר#Y59M | מספרים |
| algebraic species/number (algebraic context) | ס.פ.ר./מספר | term | חשבון_השטחים#yWsD | מספרים |
| algebraic species/number (algebraic context) | term | ספר_האלזיברא#1xDQ | אחדי המספר | |
| algebraic species/number (algebraic context) | נומרי | term | ספר_האלזיברא#Ig06 | נוּמְרִי |
| algebraic species/number (algebraic context) | ס.פ.ר./מספר | definition | אגרת_המספר#8t9R | the constants are any number whether of the units, tens, or hundreds, or the rest of the ranks, or a combination of them; be it a large number or a small number; such as: 5, 9, 11, 120; and in general: any number huge or small; therefore this rank is called by the name of the number. המספרים הם כל מספר שיהיה מן האחדים או העשרות או המאות או שאר המדרגות או מהרכבתם גדול או קטן כמו ה' וט' וי"א ק"כ ובכלל כל מספר רב או מעט ובעבור זה קראו זאת המדרגה בשם המספר |
| algebraic species/number (algebraic context) | ס.פ.ר./מספר | term | אגרת_המספר#IeqF | מספר |
| algebraic species/number (algebraic context) | ס.פ.ר./מספר | term | אגרת_המספר#Snio | מספרים |
| algebraic species/number (algebraic context) | דרהם | term | תחבולות_המספר#s8uc | אדרהמי |
| algebraic species/number (algebraic context) | דרהם | term | תחבולות_המספר#ViGL | דרהמי |
| algebraic species/number (algebraic context) | term | חשבון_השטחים#QWuq | קונטאש | |
| algebraic species/number (algebraic context) | דרהם | term | חשבון_השטחים#TKtR | אדרהמיש |
| algebraic species/number (algebraic context) | דרהם | term | חשבון_השטחים#NYjU | דרהמיש |
| algebraic species/number (algebraic context) | דרהם | term | חשבון_השטחים#QIOD | דרהם |
| algebraic species/number (algebraic context) | ס.פ.ר./מספר | term | ספר_ג'יבלי_אלמוקבאלא#dADw | מספר |
| algebraic species/number (algebraic context) | דרהם | term | ספר_ג'יבלי_אלמוקבאלא#jOOp | דראמא |
| algebraic species/number (algebraic context) | definition | תחבולות_המספר#ylJs | The number is the number which cannot be understood as a root nor a square, but is related to itself only by the units comprised in it. המספר הוא {{#annot: term | #number, #מנין | n8lE}}המנין{{#annotend:n8lE}} שלא יובנו בו שיהיה לא שרש ולא מרובע אבל הוא {{#annot: term | #to relate, #נערך | qQmi}}נערך לעצמו{{#annotend:qQmi}} במה שבו מן האחדים לבד | |
| algebraic species/number (algebraic context) | definition | חשבון_השטחים#Oq7f | The number is what increases by itself, which cannot be named a root nor a square and is related to the units comprised in it. ו{{#annot:term|35,1434|uzyY}}המנין{{#annotend:uzyY}} הוא {{#annot:term|1053,2027|HEX5}}הצומח{{#annotend:HEX5}} מאליו אשר לא יכל ליפול בו לא שם שורש ולא שם אלגו והוא {{#annot:term|1567,2586|pz9P}}המתיחס אל {{#annotend:pz9P}}מה שבו מן האחדים | |
| equation/quadratic equation | 3√x²+4√(x²-3√x²)=20 | חשבון_השטחים#tZ8i | [13] If you are told: three roots of the square plus four roots of what remains from the square are twenty dirham.
: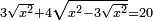 ואם יאמרו לך שלשה שרשים מהאלגו וארבעה שרשים ממה שנשאר מהאלגו יהיה עשרים דרהמי'
ואם יאמרו לך שלשה שרשים מהאלגו וארבעה שרשים ממה שנשאר מהאלגו יהיה עשרים דרהמי' | |
| equation/quadratic equation | [x²+√(x²)+√(½x²)]²=5x² | חשבון_השטחים#omoo | If you are told: a square, add to it its root and a root of its half, then multiply the result by itself; it is five times the square.
: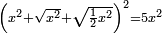 ואם יאמרו לך אלגו תוסיף עליו שרשו ושרש חציו ותכה המקובץ על עצמו ויהיה חמשה דמיוני האלגו
ואם יאמרו לך אלגו תוסיף עליו שרשו ושרש חציו ותכה המקובץ על עצמו ויהיה חמשה דמיוני האלגו | |
| equation/quadratic equation | x²+4√(x²)+√(½x²)+√(⅓x²)=10 | חשבון_השטחים#b04G | If you are told: a square, add to it its four roots plus a root of its half and a root of its third; it is ten dirham - how much is the square?
: ואם יאמר לך אלגו תוסיף עליו ארבעה שרשיו ושרש חציו ושרש שלישיתו ויהיה עשרה דרהמי כמה הוא האלגו
ואם יאמר לך אלגו תוסיף עליו ארבעה שרשיו ושרש חציו ושרש שלישיתו ויהיה עשרה דרהמי כמה הוא האלגו | |
| equation/quadratic equation | c=³√ax² | ספר_ג'יבלי_אלמוקבאלא#zK2y | When numbers are equal to a cube root of squares:
:![\scriptstyle c=\sqrt[3]{ax^2}](/mediawiki/images/math/f/7/6/f7617468fb35452327a7d2d5678e45f0.png) כאשר המספרי' יהיו שוים אל שרשי' מעו' מצינסי
כאשר המספרי' יהיו שוים אל שרשי' מעו' מצינסי | |
| equation/quadratic equation | 2√(x²)+√(½x²)+√(⅓x²)=x² | חשבון_השטחים#nPTX | If you are told: a square whose two roots plus a root of half the square and a root of its third are equal to the square - how much is the square?
: ואם יאמרו לך אלגו אשר שני שרשיו ושרש חצי האלגו ושרש שלישיתו ישוו האלגו כמה הוא האלגו
ואם יאמרו לך אלגו אשר שני שרשיו ושרש חצי האלגו ושרש שלישיתו ישוו האלגו כמה הוא האלגו | |
| equation/quadratic equation | [x²+√(x²)+√(½x²)]²=20 | חשבון_השטחים#OX0E | 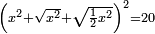 ואם יאמרו לך אלגו הוסף עליו שרשו ושרש חציו ותכה המקובץ בעצמו ויהיה עשרים דרהמי
ואם יאמרו לך אלגו הוסף עליו שרשו ושרש חציו ותכה המקובץ בעצמו ויהיה עשרים דרהמי | |
| equation/quadratic equation | x²·(x²+√10)=9x² | חשבון_השטחים#XpMD | [32] If you are told: a square, multiply it by itself plus a root of ten dirham and it becomes nine times the square.
: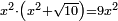 ואם יאמרו לך אלגו תכהו על עצמו ושרש אחד מעשרה דרהמי ויהיה תשעה דמיוני האלגו
ואם יאמרו לך אלגו תכהו על עצמו ושרש אחד מעשרה דרהמי ויהיה תשעה דמיוני האלגו | |
| equation/quadratic equation | ax²=³√c | ספר_ג'יבלי_אלמוקבאלא#rGD5 | When squares are equal to a cube root of the numbers:
:![\scriptstyle ax^2=\sqrt[3]{c}](/mediawiki/images/math/f/c/0/fc0033e1c190540235d4afbc47b31b11.png) כאשר הצינסי יהיו שוים אל שרשי' מעו' ממספרי'
כאשר הצינסי יהיו שוים אל שרשי' מעו' ממספרי' | |
| equation/quadratic equation | [√(x²·2x²)+2]·x²=30 | חשבון_השטחים#uwLQ | [40] If you are told: a square, you multiply it by its two times, extract the root of the product, add two dirham to it, then multiply the total by that square and the result is thirty dirham.
: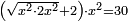 ואם יאמרו לך אלגו תכהו על {{#annot:term|387|piMO}}שני דמיוניו{{#annotend:piMO}} ותקח שרש העולה ותוסיף עליו שני דרהמי ותכה הכל באלגו ההוא ויהיה שלשים דרהמי
ואם יאמרו לך אלגו תכהו על {{#annot:term|387|piMO}}שני דמיוניו{{#annotend:piMO}} ותקח שרש העולה ותוסיף עליו שני דרהמי ותכה הכל באלגו ההוא ויהיה שלשים דרהמי | |
| equation/quadratic equation | [x²+√(½x²)]²=4x² | חשבון_השטחים#Fvgh | If you are told: a square, you add to it a root of its half, then multiply the result by itself; it becomes four times the square.
: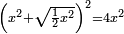 ואם יאמרו לך אלגו הוספת עליו שרש חציו והכית העולה בעצמו ויהיה ארבעה דמיוני האלגו
ואם יאמרו לך אלגו הוספת עליו שרש חציו והכית העולה בעצמו ויהיה ארבעה דמיוני האלגו | |
| equation/quadratic equation | (√10·x²)/(2√3)=x²-10 | חשבון_השטחים#8OnP | If you are told: a square, multiply it by the root of ten, then divide the product by two plus the root of three; the quotient is the same as the square minus ten.
: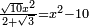 ואם יאמרו לך אלגו תכהו בשרש עשרה ותחלק מה שיעלה על שנים ושרש שלשה ויעלה לאחד כל כך כמו שהוא האלגו פחות עשרה
ואם יאמרו לך אלגו תכהו בשרש עשרה ותחלק מה שיעלה על שנים ושרש שלשה ויעלה לאחד כל כך כמו שהוא האלגו פחות עשרה | |
| equation/quadratic equation | (x²+10)·√5=(x²)² | חשבון_השטחים#hePu | [38] If you are told: a square, you add to it ten dirham, then multiply the sum by a root of five and the result is the same as the product of the square by itself.
: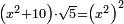 ואם יאמרו לך אלגו הוספת עליו עשרה דרהמי והכית המקובץ בשרש חמשה ויעלה כמו הכאת האלגו על עצמו
ואם יאמרו לך אלגו הוספת עליו עשרה דרהמי והכית המקובץ בשרש חמשה ויעלה כמו הכאת האלגו על עצמו | |
| equation/quadratic equation | (x²+7)·√(3x²)=10x² | חשבון_השטחים#dAZJ | If you are told: a square, add to it seven dirham, then multiply the sum by a root of three times the square; it becomes ten times the square.
: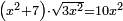 ואם יאמרו לך אלגו תוסיף עליו שבעה דרהמי ותכה המקובץ בשרש שלשה דמיוני האלגו ויהיה עשרה דמיוני האלגו
ואם יאמרו לך אלגו תוסיף עליו שבעה דרהמי ותכה המקובץ בשרש שלשה דמיוני האלגו ויהיה עשרה דמיוני האלגו | |
| equation/quadratic equation | 3√x²+4√(x²-3√x²)=x²+4 | חשבון_השטחים#SNBo | [17] If you are told: a square, its three roots and four roots of what remains from the square are the same as the square plus four dirham.
: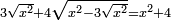 ואם יאמרו לך אלגו שלשת שרשיו וארבעה שרשים ממה שנשאר מהאלגו יהיה כמו האלגו וארבעה דרהמי' יותר
ואם יאמרו לך אלגו שלשת שרשיו וארבעה שרשים ממה שנשאר מהאלגו יהיה כמו האלגו וארבעה דרהמי' יותר | |
| equation/quadratic equation | 3√x²+2√(x²-3√x²)=x² | חשבון_השטחים#rq9x | [16] If you are told: a square, its three roots and two roots of what remains are equal to the square.
: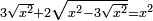 ואם יאמרו לך אלגו שלשת שרשיו ושני שרשי הנשאר ישוו האלגו
ואם יאמרו לך אלגו שלשת שרשיו ושני שרשי הנשאר ישוו האלגו | |
| equation/quadratic equation | (x²-⅓x²)·3√x²=x² | חשבון_השטחים#ak8r | [14] If you are told: we subtract from a square its third, then multiply what remains by three roots of the original square and the result is the original square.
: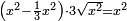 ואם יאמרו לך גרענו מן האלגו שלישיתו והכינו הנשאר על שלשה שרשים מהאלגו הראשון ושב האלגו הראשון
ואם יאמרו לך גרענו מן האלגו שלישיתו והכינו הנשאר על שלשה שרשים מהאלגו הראשון ושב האלגו הראשון | |
| equation/quadratic equation | [x²-(⅓x²+2)]²=x²+24 | חשבון_השטחים#lT08 | [12] If you are told: a square, you subtract its third and two dirham from it, then multiply what remains by itself and the result is the square plus 24 dirham.
:![\scriptstyle\left[x^2-\left(\frac{1}{3}x^2+2\right)\right]^2=x^2+24](/mediawiki/images/math/a/9/5/a957ce494441269eddb2735f8c5cdf54.png) ואם יאמרו לך אלגו גרעת ממנו שלישיתו ושני אדרהמיש ומה שנשאר הכית אותו על עצמו ושב האלגו וכ"ד אדרהמיש יותר
ואם יאמרו לך אלגו גרעת ממנו שלישיתו ושני אדרהמיש ומה שנשאר הכית אותו על עצמו ושב האלגו וכ"ד אדרהמיש יותר | |
| equation/quadratic equation | [x²+√(3x²)]·√(2x²)=4x² | חשבון_השטחים#lk6C | If you are told: a square, add to it a root of three times of it, then multiply the sum by a root of [twice] the square; it becomes four times the square.
: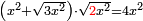 ואם יאמרו לך אלגו תוסיף עליו שרש שלשה דמיוניו ותכה המקובץ בשרש האלגו יהיה ארבעה דמיוני האלגו
ואם יאמרו לך אלגו תוסיף עליו שרש שלשה דמיוניו ותכה המקובץ בשרש האלגו יהיה ארבעה דמיוני האלגו | |
| equation/quadratic equation | [√(6x²)·√(5x²)]+10x²+20=(x²)² | חשבון_השטחים#stdc | [34] If you are told: a square, multiply a root of its six times by a root of its five times, then add ten times the square plus twenty dirham to the product and it is the same as [the product of] the square by itself.
: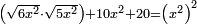 ואם יאמרו לך אלגו תרבה שרש מששה דמיוניו בשרש חמשה דמיוניו והוספת על מה שעלה מההכאה עשרה דמיוני האלגו ועשרים דרהמי יותר ויהיה כמו האלגו על עצמו
ואם יאמרו לך אלגו תרבה שרש מששה דמיוניו בשרש חמשה דמיוניו והוספת על מה שעלה מההכאה עשרה דמיוני האלגו ועשרים דרהמי יותר ויהיה כמו האלגו על עצמו | |
| equation/quadratic equation | [√(8x²)·√(3x²)]+20=(x²)² | חשבון_השטחים#HcHI | [33] If you are told: a square, multiply a root of eight times the square by a root of three times the square, then add twenty dirham to the result and it is the same as [the product of] the square by itself.
: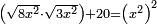 ואם יאמרו לך אלגו תרבה שרש משמנה דמיוני האלגו בשרש משלשה דמיוני האלגו והוספת על העולה עשרים דרהמי ויהיה כמו האלגו על עצמו
ואם יאמרו לך אלגו תרבה שרש משמנה דמיוני האלגו בשרש משלשה דמיוני האלגו והוספת על העולה עשרים דרהמי ויהיה כמו האלגו על עצמו | |
| equation/quadratic equation | (x²-⅓x²)·3√(x²-⅓x²)=x² | חשבון_השטחים#lFM5 | [15] If you are told: a square, we subtract its third from it, then multiply what remains by three roots of what remains from the square and the result is the square.
: ואם יאמרו לך אלגו גרענו ממנו שלישיתו והכינו הנשאר על שלשה שרשים מהנשאר מהאלגו וישוב האלגו
ואם יאמרו לך אלגו גרענו ממנו שלישיתו והכינו הנשאר על שלשה שרשים מהנשאר מהאלגו וישוב האלגו | |
| equation/quadratic equation | a²+b²=c², ac=b², ab=10 | חשבון_השטחים#gGKe | If you are told: three unequal squares, if you multiply the smaller by itself and the mean by itself; they are as the greater by itself. If you multiply the smaller by the greater it is as the mean by itself. If you multiply the smaller by the mean it is ten dirham.
: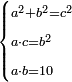 ואם יאמרו לך שלשה אלגוש בלתי שוים
ואם יאמרו לך שלשה אלגוש בלתי שויםאם תכה הקטן בעצמו והאמצעי בעצמו יהיו כמו הגדול בעצמו ואם תכה הקטון בגדול יהיה כמו האמצעי בעצמו ואם תכה הקטן באמצעי יהיה עשרה דרהמי | |
| equation/quadratic equation | [x²-(2√x²+10)]²=8x² | חשבון_השטחים#aPQX | If you are told: a square, subtract its two roots and ten dirham from it, then multiply what remains by itself; it becomes eight times the square.
:![\scriptstyle\left[x^2-\left(2\sqrt{x^2}+10\right)\right]^2=8x^2](/mediawiki/images/math/1/4/5/145c5c99f9bc23a17e6ee34a2c9df452.png) ואם יאמרו לך אלגו תגרע ממנו שני שרשיו ועשרה דרהמי ותכה מה שישאר בעצמו ויהיה שמנה דמיוני האלגו
ואם יאמרו לך אלגו תגרע ממנו שני שרשיו ועשרה דרהמי ותכה מה שישאר בעצמו ויהיה שמנה דמיוני האלגו | |
| equation/quadratic equation | b²=3a², (a²+√a²)·(b²+√b²)=10b² | חשבון_השטחים#nHFm | If you are told: two squares - one is three times the other; you add to each of them its root, then multiply the one by the other; it is ten times the greater square.
: ואם יאמרו שני אלגוש והאחד שלשה דמיוני האחר הוספת על כל אחד מהם שרשו שלו והכית האחד באחר והיה עשרה דמיוני האלגו הגדול
ואם יאמרו שני אלגוש והאחד שלשה דמיוני האחר הוספת על כל אחד מהם שרשו שלו והכית האחד באחר והיה עשרה דמיוני האלגו הגדול | |
| equation/quadratic equation | √x²+√(√x²)+√(2√x²)+5√x²=10 | חשבון_השטחים#rWIe | If you are told: a square whose root and the root of its root, plus the root of its two roots, plus the root of five times the square are ten dirham.
: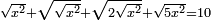 ואם יאמרו לך אלגו אשר שרשו ו{{#annot:term|2634|dE2e}}שרש שרשו{{#annotend:dE2e}} ושרש שני שרשיו ושרש חמשה דמיוני האלגו יהיה עשרה דרהמי
ואם יאמרו לך אלגו אשר שרשו ו{{#annot:term|2634|dE2e}}שרש שרשו{{#annotend:dE2e}} ושרש שני שרשיו ושרש חמשה דמיוני האלגו יהיה עשרה דרהמי | |
| equation/quadratic equation | 2√(x²)+√(½x²)+√(⅓x²)=20 | חשבון_השטחים#wOZV | If one says: a square whose two roots plus a root of its half and a root of its third are twenty dirham - how much is the square?
: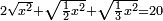 ואם יאמר אלגו אשר שני שרשיו ושרש חציו ושרש שלישיתו עשרים דרהמי כמה הוא האלגו
ואם יאמר אלגו אשר שני שרשיו ושרש חציו ושרש שלישיתו עשרים דרהמי כמה הוא האלגו | |
| equation/quadratic equation | [√(½x²)+3]·[√(⅓x²)+2]=20 | חשבון_השטחים#Y7kO | If you are told: a square, add three dirham to a root of its half, and two dirham to a root of its third, then multiply one [sum] by the other; it is twenty dirham.
: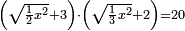 ואם יאמרו לך אלגו תוסיף על שרש חציו שלשה דרהמי ועל שרש שלישיתו שני דרהמי ותכה האחד באחר ויהיה עשרים דרהמי
ואם יאמרו לך אלגו תוסיף על שרש חציו שלשה דרהמי ועל שרש שלישיתו שני דרהמי ותכה האחד באחר ויהיה עשרים דרהמי | |
| algebraic operation/restoration | אלג'בר | term | קצת_מענייני_חכמת_המספר#ul5O | ג'בר |
| algebraic operation/restoration | term | תחבולות_המספר#bYhV | קובראמיינטו | |
| algebraic operation/restoration | ש.ל.מ./השלמה | term | אגרת_המספר#DMPJ | השלמה |
| algebraic operation/restoration | פ.ק.ד./הפקדה | term | תחבולות_המספר#vlNP | הפקדה |
| algebraic operation/restoration | term | חשבון_השטחים#udFo | קובראמיינטו | |
| algebraic operation/restoration | אלג'בר | term | ספר_החשבון_לאל_חצאר#Oarc | באלגבר |
| compound canonical equation/roots and numbers equal squares | 3x+4=x² | ספר_המספר_/_אליהו_מזרחי#7D6K | Question: if one asks: what is the square such that 3 times its root plus 4 equals 10.
: שאלה אם שאל שואל איזהו המרובע אשר ג' כפלי שרשו וד' ישוו לי'
שאלה אם שאל שואל איזהו המרובע אשר ג' כפלי שרשו וד' ישוו לי' | |
| compound canonical equation/roots and numbers equal squares | 3x+4=x² | תחבולות_המספר#VKnl | He said: roots and numbers that are equal to a square is as saying three roots and four dirham are equal to a square.
: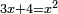 אמ' שרשים ומספרים שישוו למרובע הוא כמו שיאמ' שלשה שרשים וארבעה דרהמי יהיו שוים למרובע
אמ' שרשים ומספרים שישוו למרובע הוא כמו שיאמ' שלשה שרשים וארבעה דרהמי יהיו שוים למרובע | |
| compound canonical equation/roots and numbers equal squares | bx+c=ax² | חשבון_השטחים#VBed | ושרשים ומספרי' ישוו למרובעים | |
| compound canonical equation/roots and numbers equal squares | bx+c=ax² | ספר_האלזיברא#zm1d | הדברים והאחדים שוים למרובעים | |
| compound canonical equation/roots and numbers equal squares | bx+c=ax² | תחבולות_המספר#So10 | ושרשי' ומספרים שישוו למרובעים | |
| compound canonical equation/roots and numbers equal squares | 3x+4=x² | חשבון_השטחים#qrpb | 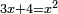 והשרשים ומספרים שישוו אלגוש כמו אם יאמר שלשה שרשים וארבעה מספרים ישוו אלגו
והשרשים ומספרים שישוו אלגוש כמו אם יאמר שלשה שרשים וארבעה מספרים ישוו אלגו | |
| compound canonical equation/roots and numbers equal squares | 3x+4=x² | ספר_ג'יבלי_אלמוקבאלא#GUT6 | For example, suppose that 3 things and 4 numbers are equal to 1 square.
::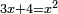 והנה המשל נניח כי שלשה דברי' וד' דרמי רצוני ד' מספרי' יהיו שוים אל א' צינסו
והנה המשל נניח כי שלשה דברי' וד' דרמי רצוני ד' מספרי' יהיו שוים אל א' צינסו | |
| simple canonical equation/roots equal numbers | bx=c | חשבון_השטחים#1nZH | ושרשים ישוו מספרים | |
| simple canonical equation/roots equal numbers | ½x=10 | חשבון_השטחים#0Ojb | If you say: half a root is equal to ten.
: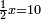 וכמו אם תאמר חצי שרש ישוה עשרה
וכמו אם תאמר חצי שרש ישוה עשרה | |
| simple canonical equation/roots equal numbers | x=4 | תחבולות_המספר#PaMj | If you are told: the roots of the square are equal to four numbers.
: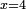 כי אם יאמרו לך שרשי המרובע ישוו לארבע' מספרי'
כי אם יאמרו לך שרשי המרובע ישוו לארבע' מספרי' | |
| simple canonical equation/roots equal numbers | ½x=10 | תחבולות_המספר#e4m4 | If one says: half a root is equal to ten.
: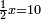 ואם אמ'
ואם אמ' | |
| simple canonical equation/roots equal numbers | 5x=30 | חשבון_השטחים#1YGh | Also if you say: five roots are equal to thirty.
: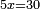 וכמו אם תאמר חמשה שרשים ישוו שלשים
וכמו אם תאמר חמשה שרשים ישוו שלשים | |
| simple canonical equation/roots equal numbers | 5x=30 | תחבולות_המספר#jHg9 | As if one says: five roots are equal to thirty.
: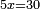 כמו אם אמ' חמשה שרשים ישוו לשלשים
כמו אם אמ' חמשה שרשים ישוו לשלשים | |
| simple canonical equation/roots equal numbers | bx=c | תחבולות_המספר#qsBh | ושרשים שישוו למספרים | |
| simple canonical equation/roots equal numbers | x=4 | חשבון_השטחים#wMvn | When the roots are equal to numbers, as if you say: the root is equal to four.
: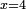 והשרשים שישוו מספרים כמו שתאמר שרש ישוה ארבעה
והשרשים שישוו מספרים כמו שתאמר שרש ישוה ארבעה | |
| simple canonical equation/roots equal numbers | bx=c | ספר_האלזיברא#uues | הדברים שוים לאחדים | |
| equation/side of an equation | term | ספר_האלזיברא#zNHv | החלקים | |
| canonical equation/simple canonical equation | פ.ר.ד./נפרד | term | חשבון_השטחים#o3Jf | נפרדים |
| canonical equation/simple canonical equation | פ.ש.ט./פשוט | term | תחבולות_המספר#Zvsu | הפשוטים |
| canonical equation/simple canonical equation | definition | חשבון_השטחים#Ja1c | הנה השלשה מהם {{#annot: term | #simple canonical equation, #נפרד | o3Jf}}נפרדים{{#annotend:o3Jf}} והם אלגוש ישוו שרשים והאלגו ישוו מספרים ושרשים ישוו מספרים | |
| compound canonical equation/squares and numbers equal roots | ax²+c=bx | ספר_האלזיברא#oeK8 | המרובעים והאחדים שוים לדברים | |
| compound canonical equation/squares and numbers equal roots | 3x²+21=10x | ספר_המספר_/_אליהו_מזרחי#3RbO | As, if you say: what is the square whose product by 3 plus 21 equals 10 times its root.
: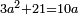 כמו שתאמר איזהו המרובע אשר ג' כפליו עם כ"א ישוו לי' כפלי שרשו
כמו שתאמר איזהו המרובע אשר ג' כפליו עם כ"א ישוו לי' כפלי שרשו | |
| compound canonical equation/squares and numbers equal roots | x²+21=10x | ספר_המספר_/_אליהו_מזרחי#hfpC | Question: if one asks: what is the square whose sum with twenty-one, for instance, yields the same as ten times its root.
: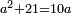 שאלה אם שאל שואל איזהו המרובע אשר חבורו עם עשרים ואחד על דרך משל יעלה כמו עשרה כפלי שרשו
שאלה אם שאל שואל איזהו המרובע אשר חבורו עם עשרים ואחד על דרך משל יעלה כמו עשרה כפלי שרשו | |
| compound canonical equation/squares and numbers equal roots | ⅓x²+21=10x | ספר_המספר_/_אליהו_מזרחי#DMJh | As, if you say: what is the square whose third plus 21 equals 10 times its root.
: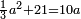 כמו שתאמר איזהו המרובע אשר שלישתו עם כ"א ישוו לי' כפלי שרשו
כמו שתאמר איזהו המרובע אשר שלישתו עם כ"א ישוו לי' כפלי שרשו | |
| compound canonical equation/squares and numbers equal roots | x²+25=10x | חשבון_השטחים#5rN4 | 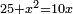 ו{{#annot: term | #to give an example, #משל | ZpYe}}אמשיל זה{{#annotend:ZpYe}} בשאילה אחרת ואניחנה שכ"ה אדרהמיש ואלגוש ישוו עשרה שרשים
ו{{#annot: term | #to give an example, #משל | ZpYe}}אמשיל זה{{#annotend:ZpYe}} בשאילה אחרת ואניחנה שכ"ה אדרהמיש ואלגוש ישוו עשרה שרשים | |
| compound canonical equation/squares and numbers equal roots | ax²+c=bx | חשבון_השטחים#iECf | ומרובעים ומספרי' ישוו לשרשים | |
| compound canonical equation/squares and numbers equal roots | x²+25=10x | תחבולות_המספר#QWm3 | Example: one says: a square plus twenty-five [dirham] are equal to ten roots of the square.
: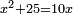 דמיון זה שיאמ' מרובע מה ועשרים וחמשה שרשים ישוו לעשרה שרשים מהמרובע
דמיון זה שיאמ' מרובע מה ועשרים וחמשה שרשים ישוו לעשרה שרשים מהמרובע | |
| compound canonical equation/squares and numbers equal roots | x²+21=10x | חשבון_השטחים#yRRf | 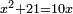 והאלגוש והמספרים שישוו שרשים כאלו תאמר אלגוש ועשרים ואחד אדרהמיש ישוו עשרה שרשים
והאלגוש והמספרים שישוו שרשים כאלו תאמר אלגוש ועשרים ואחד אדרהמיש ישוו עשרה שרשים | |
| compound canonical equation/squares and numbers equal roots | ax²+c=bx | תחבולות_המספר#om8w | ומרובעי' ומספרי' שישוו לשרשים | |
| compound canonical equation/squares and numbers equal roots | x²+21=10x | תחבולות_המספר#u4EA | He said: squares and numbers that are equal to roots is as if you say: when you sum twenty-one dirham with a certain square, they are equal to ten roots of the square.
: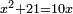 אמ' המרובעי' והמספרי' שישוו לשרשים הוא כאלו תאמ' כאשר תקבץ עם מרובע מה עשרים ואחד דרהמי יהיו שוים לעשרה משרשים מהמרובע
אמ' המרובעי' והמספרי' שישוו לשרשים הוא כאלו תאמ' כאשר תקבץ עם מרובע מה עשרים ואחד דרהמי יהיו שוים לעשרה משרשים מהמרובע | |
| compound canonical equation/squares and numbers equal roots | x²+21=10x | ספר_ג'יבלי_אלמוקבאלא#RpEs | You get one square and 21 numbers equal 10 things.
::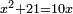 ויהיה לך א' צינסו וכ"א מספרי' שוים לי' דברי'
ויהיה לך א' צינסו וכ"א מספרי' שוים לי' דברי' | |
| compound canonical equation/squares and roots equal numbers | x²+10x=39 | ספר_המספר_/_אליהו_מזרחי#efjm | Question: if one asks: what is the square whose sum with ten times its root, for instance, yields thirty-nine.
: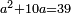 שאלה אם ישאל שואל איזהו המרובע אשר חבורו עם עשר כפלי שרשו על דרך משל יעלו תשעה ושלשים
שאלה אם ישאל שואל איזהו המרובע אשר חבורו עם עשר כפלי שרשו על דרך משל יעלו תשעה ושלשים | |
| compound canonical equation/squares and roots equal numbers | ax²+bx=c | ספר_האלזיברא#vJ0S | המרבעים והדברי' שוים לאחדים | |
| compound canonical equation/squares and roots equal numbers | x²+10x=39 | ספר_ג'יבלי_אלמוקבאלא#jJWT | 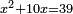 ויהיה לך צינסו אחד וי' דברי' שוים לל"ט
ויהיה לך צינסו אחד וי' דברי' שוים לל"ט | |
| compound canonical equation/squares and roots equal numbers | ax²+bx=c | חשבון_השטחים#XqGp | מרובעי' ושרשי' ישוו למספרים | |
| compound canonical equation/squares and roots equal numbers | x²+10x=39 | תחבולות_המספר#oMPa | He said: when squares and roots are equal to numbers, it is as if you say: the sum of one square and ten of its roots together is equal to thirty-nine dirham.
: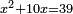 אמ' כאשר יהיו המרובעים והשרשים שוים למספרי' כאלו תאמ' המקובץ ממרובע מהאחד ועשרה משרשיו יחד ישוה לשלשים ותשעה דרהמי
אמ' כאשר יהיו המרובעים והשרשים שוים למספרי' כאלו תאמ' המקובץ ממרובע מהאחד ועשרה משרשיו יחד ישוה לשלשים ותשעה דרהמי | |
| compound canonical equation/squares and roots equal numbers | ⅓x²+3x=30 | ספר_המספר_/_אליהו_מזרחי#109r | As, for example, if one asks: what is the square whose third plus 12 times its root are thirty.
: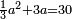 כמו על דרך משל אם שאל שואל איזהו המרובע אשר שלישיתו עם ג' כפלי שרשו הם שלשים
כמו על דרך משל אם שאל שואל איזהו המרובע אשר שלישיתו עם ג' כפלי שרשו הם שלשים | |
| compound canonical equation/squares and roots equal numbers | ax²+bx=c | תחבולות_המספר#3sJZ | ושרשים ומרובעי' שישוו למספרים | |
| compound canonical equation/squares and roots equal numbers | x²+10x=39 | ספר_המספר_/_אליהו_מזרחי#5Cr3 | The example in the mentioned question: one square plus ten times its root are thirty-nine.
: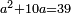 המשל בזה בשאלה הנזכרת והיא שהמרובע הא' עם עשרה כפלי שרשו הם תשעה ושלשים
המשל בזה בשאלה הנזכרת והיא שהמרובע הא' עם עשרה כפלי שרשו הם תשעה ושלשים | |
| compound canonical equation/squares and roots equal numbers | ½x²+5x=28 | חשבון_השטחים#XyaI | 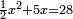 ואם אמרו לך חצי אלגוש וחמשה שרשים ישוו כ"ח אדרהמיש
ואם אמרו לך חצי אלגוש וחמשה שרשים ישוו כ"ח אדרהמישנאמר כי כאשר {{#annot: term | #to add, #חבר | qgPe}}חברנו{{#annotend:qgPe}} על חצי האלגוש חמשה שרשי האלגוש יהיו כ"ח אדרהמיש | |
| compound canonical equation/squares and roots equal numbers | ½x²+5x=28 | תחבולות_המספר#FJRf | Likewise, if one asks: half a square plus its five roots are equal to 28 dirham.
: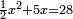 וכמו כן אם שאל השואל חצי מרובע וחמשה משרשיו ישוו לכ"ח דרהמי
וכמו כן אם שאל השואל חצי מרובע וחמשה משרשיו ישוו לכ"ח דרהמי | |
| compound canonical equation/squares and roots equal numbers | 3x²+15x=72 | תחבולות_המספר#HV5b | Example: if one asks: three squares and 15 roots are equal to 72 dirham.
: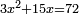 דמיון זה ששאל שלשה מרובעי' וט"ו שרשי' ישוו לע"ב דרהמי
דמיון זה ששאל שלשה מרובעי' וט"ו שרשי' ישוו לע"ב דרהמי | |
| compound canonical equation/squares and roots equal numbers | 2x²+10x=48 | חשבון_השטחים#cSpR | 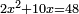 וזה כמו שתאמר שנים אלגוש ועשרה שרשים 2rישוו מ"ח אדרהמיש
וזה כמו שתאמר שנים אלגוש ועשרה שרשים 2rישוו מ"ח אדרהמיש | |
| compound canonical equation/squares and roots equal numbers | x²+10x=39 | חשבון_השטחים#BnUp | Squares and roots are equal to numbers, it is as if you say: a square and ten roots are equal to thirty-nine dirham.
: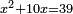 והאלגוש והשרשים שישוו מספרים הוא כאלו תאמר אלגו ועשרה שרשים ישוו תשעה ושלשים דרהמיש
והאלגוש והשרשים שישוו מספרים הוא כאלו תאמר אלגו ועשרה שרשים ישוו תשעה ושלשים דרהמיש | |
| compound canonical equation/squares and roots equal numbers | 3x²+12x=180 | ספר_המספר_/_אליהו_מזרחי#egqO | As, for example, if one asks: what is the square whose product by 3 plus 12 times its root, yields 180.
: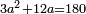 כמו על דרך משל אם שאל שואל איזהו המרובע אשר ג' כפליו עם י"ב כפלי שרשו יעלו ק"פ
כמו על דרך משל אם שאל שואל איזהו המרובע אשר ג' כפליו עם י"ב כפלי שרשו יעלו ק"פ | |
| simple canonical equation/squares equal numbers | x²=16 | תחבולות_המספר#fCC4 | As if you are told: the square is equal to sixteen.
: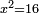 כמו אם יאמרו לך המרובע ישוה לששה עשר
כמו אם יאמרו לך המרובע ישוה לששה עשר | |
| simple canonical equation/squares equal numbers | ax²=c | חשבון_השטחים#kCXF | ואלאגוש ישוו מספרים | |
| simple canonical equation/squares equal numbers | ⅓x²=27 | חשבון_השטחים#sOLH | Also if you say: a third of the square is equal to twenty-seven.
: וכן אם תאמר שלישית אלגו שוה 1vשבעה ועשרים
וכן אם תאמר שלישית אלגו שוה 1vשבעה ועשרים | |
| simple canonical equation/squares equal numbers | 5x²=45 | חשבון_השטחים#yMV8 | Likewise, when five squares are equal to forty-five.
: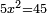 וכן חמשה אלגוש ישוו חמשה וארבעים
וכן חמשה אלגוש ישוו חמשה וארבעים | |
| simple canonical equation/squares equal numbers | ⅓x²=27 | תחבולות_המספר#Usmi | If one says: a third of the square is equal to twenty-seven.
: ואם אמ' שלישית המרובע ישוה לשבעה ועשרים
ואם אמ' שלישית המרובע ישוה לשבעה ועשרים | |
| simple canonical equation/squares equal numbers | 5x²=45 | תחבולות_המספר#C4DX | If one says: five squares are equal to forty-five.
: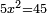 כי אם אמ' חמשה מרובעי' ישוו לחמשה וארבעי'
כי אם אמ' חמשה מרובעי' ישוו לחמשה וארבעי' | |
| simple canonical equation/squares equal numbers | ax²=c | ספר_האלזיברא#jx5h | המרבעים צינסי שוים לאחדים | |
| simple canonical equation/squares equal numbers | x²=16 | חשבון_השטחים#lI7w | The squares that are equal to numbers is as a square that equals sixteen.
: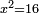 והאלגוש שישוו מספרים כמו אלגו שישוה שש עשרה
והאלגוש שישוו מספרים כמו אלגו שישוה שש עשרה | |
| simple canonical equation/squares equal numbers | ax²=c | תחבולות_המספר#4O9C | ומרובעי' שישוו למספרים | |
| simple canonical equation/squares equal roots | ½x²=10x | חשבון_השטחים#N1ie | Likewise, if it is said: half a square equals ten roots.
: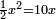 וכמו כן אם יאמר חצי אלגו ישוה עשרה שרשים
וכמו כן אם יאמר חצי אלגו ישוה עשרה שרשים | |
| simple canonical equation/squares equal roots | 5x²=20x | חשבון_השטחים#sJUh | As if you say: five squares equal twenty roots.
: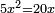 וכמו שתאמר חמשה אלגוש ישוו עשרים שרשים
וכמו שתאמר חמשה אלגוש ישוו עשרים שרשים | |
| simple canonical equation/squares equal roots | ½x²=10x | תחבולות_המספר#fayj | Also, if he says: half a square is equal to ten roots.
: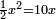 וכן אם יאמ' חצי מרובע ישוה לעשרה שרשים
וכן אם יאמ' חצי מרובע ישוה לעשרה שרשים | |
| simple canonical equation/squares equal roots | x²=5x | חשבון_השטחים#cbxX | The squares that are equal to roots is as if you say: a square equals five roots.
: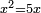 והאלגוש שישוו שרשים הוא כאלו תאמר אלגו ישוה חמשה שרשים
והאלגוש שישוו שרשים הוא כאלו תאמר אלגו ישוה חמשה שרשים | |
| simple canonical equation/squares equal roots | ax²=bx | חשבון_השטחים#bTgP | אלגוש ישוו שרשים | |
| simple canonical equation/squares equal roots | ax²=bx | תחבולות_המספר#Udbo | שרשים שיהיו שוי' למרובעים | |
| simple canonical equation/squares equal roots | 5x²=20x | תחבולות_המספר#WDhU | Example: if one asks: five squares are equal to twenty roots.
: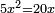 דמיון זה אם ישאל חמשה מרובעי' ישוו לעשרים שרשים
דמיון זה אם ישאל חמשה מרובעי' ישוו לעשרים שרשים | |
| simple canonical equation/squares equal roots | x²=5x | תחבולות_המספר#EqMy | For example: if you are told; five roots are equal to one square. How much is the square?
: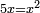 {{#annot:term|197,1712|lPkF}}דמיון זה{{#annotend:lPkF}} אם אמרו לך חמשה שרשי' ישוו למרובע אחד כמה הוא המרובע
{{#annot:term|197,1712|lPkF}}דמיון זה{{#annotend:lPkF}} אם אמרו לך חמשה שרשי' ישוו למרובע אחד כמה הוא המרובע | |
| simple canonical equation/squares equal roots | ax²=bx | ספר_האלזיברא#XXXO | המרבעים שוים לדברים | |
| confrontation/to confront | כ.ו.נ./הכוין | term | חשבון_השטחים#B9YU | תכוין |
| confrontation/to confront | ק.ב.ל./הקביל | term | ספר_החשבון_לאל_חצאר#XAbm | תקבל |
| confrontation/to confront | term | חשבון_השטחים#lYBE | איקונפרונטלוש קונילייוש | |
| confrontation/to confront | נ.כ.ח./הנכיח | term | חשבון_השטחים#OOUE | תנכח |
| confrontation/to confront | כ.ו.נ./כיוון | term | חשבון_השטחים#uZXq | תכוונהו |
| confrontation/to confront | ק.ב.ל./הקביל | term | ספר_החשבון_לאל_חצאר#0MAs | תקביל |
| confrontation/to confront | term | חשבון_השטחים#pbfK | איקונפרונטאראש | |
| confrontation/to confront | כ.ו.נ./הכוין | term | חשבון_השטחים#EjFX | יכוין |
| confrontation/to confront | term | חשבון_השטחים#FCAn | איקונפרונטלו | |
| confrontation/to confront | נ.כ.ח./הנכיח | term | חשבון_השטחים#UCpe | תנכחם |
| confrontation/to confront | כ.ו.נ./מכוון | term | חשבון_השטחים#KEfp | המכוון |
| algebraic operation/to equate | ש.ו.ה./השווה | term | ספר_האלזיברא#Tf7B | תשוה אותם |
| algebraic operation/to equate | ש.ו.ה./השווה | term | ספר_ג'יבלי_אלמוקבאלא#5GnA | השוה |
| algebraic operation/to equate | ש.ו.ה./השווה | term | ספר_ג'יבלי_אלמוקבאלא#7zzS | תשוה ל |
| algebraic operation/to equate | ש.ו.ה./משווה | term | חשבון_השטחים#uIeR | המשוים |
| algebraic operation/to equate | ש.ו.ה./השווה | term | ספר_ג'יבלי_אלמוקבאלא#zMtq | תשוה ה |
| normalization/to normalize | ש.ו.ב./השיב | term | ספר_ג'יבלי_אלמוקבאלא#RVwp | להשיבה |
| normalization/to normalize | ש.ו.ב./שב | term | חשבון_השטחים#MUVW | ישוב האלגו אל מה שהיה |
| normalization/to normalize | ש.ו.ב./השיב | term | חשבון_השטחים#tUwG | תשיבנה |
| normalization/to normalize | ח.ת.מ./החתים | term | ספר_החשבון_לאל_חצאר#9d38 | תחתים |
| normalization/to normalize | ש.ו.ב./השיב | term | חשבון_השטחים#clnS | השיבות |
| normalization/to normalize | ש.ו.ב./השיב | term | חשבון_השטחים#W1KL | השיבם אל אלגו אחד |
| normalization/to normalize | ש.ל.מ./שלם | term | חשבון_השטחים#jnM9 | ישלם |
| normalization/to normalize | term | חשבון_השטחים#zb3C | אשר ישובו אליו | |
| normalization/to normalize | ש.ו.ב./השיב | term | חשבון_השטחים#k0rT | תשיב האלגוש שלך שלם |
| normalization/to normalize | ש.ו.ב./השיב | term | תחבולות_המספר#egHC | השיבם |
| normalization/to normalize | ש.ו.ב./הושב | term | ספר_ג'יבלי_אלמוקבאלא#r7n6 | הושבה אל |
| normalization/to normalize | ש.ל.מ./השלים | term | Anonymous#PY3H | השלם |
| normalization/to normalize | ש.ו.ב./השיב | term | תחבולות_המספר#OhZb | תשיב |
| restoration/to restore | term | חשבון_השטחים#G0xQ | איקוברא | |
| restoration/to restore | א.ס.פ./אסף | term | תחבולות_המספר#wV1c | תאסוף ה |
| restoration/to restore | term | חשבון_השטחים#WDbl | איקובריאש | |
| restoration/to restore | כ.ל.ל./כלל | term | חשבון_השטחים#fGN0 | תכלול |
| restoration/to restore | י.צ.ע./הציע | term | חשבון_השטחים#Yez5 | תציעם |
| restoration/to restore | term | חשבון_השטחים#EerC | איקוברלוש | |
| restoration/to restore | כ.ל.ל./כלל | term | חשבון_השטחים#rlut | תכללם עם |
| restoration/to restore | א.ס.פ./אסף | term | חשבון_השטחים#rk4t | תאסוף |
| restoration/to restore | term | חשבון_השטחים#DA97 | איקובראראש | |
| restoration/to restore | א.ס.פ./אסף | term | חשבון_השטחים#lHjF | תאספם |
| restoration/to restore | ש.ל.מ./השלים | term | ספר_האלזיברא#HUT7 | תשלים |
| restoration/to restore | ש.ל.מ./שלם | term | ספר_ג'יבלי_אלמוקבאלא#m7fq | לשלם במקום שיחסר |
| restoration/to restore | השחית חוב | term | ספר_ג'יבלי_אלמוקבאלא#g7ov | להשחית החוב |
| restoration/to restore | ש.ל.מ./שלם | term | ספר_ג'יבלי_אלמוקבאלא#52ZN | לשלם במקום החסר |
| transformation between kinds/to transform between kinds | ש.ו.ב./הושב | term | ספר_ג'יבלי_אלמוקבאלא#HBuz | הושבו אל |
| transformation between kinds/to transform between kinds | ש.ו.ב./הושב | term | ספר_ג'יבלי_אלמוקבאלא#Ycae | מושב ל |
| transformation between kinds/to transform between kinds | ש.ו.ב./השיב | term | ספר_ג'יבלי_אלמוקבאלא#1lg4 | תשיב המספרי' לשרשי' |
| transformation between kinds/to transform between kinds | ש.ו.ב./הושב | term | ספר_ג'יבלי_אלמוקבאלא#b0jH | הושב אל |
| transformation between kinds/to transform between kinds | ש.ו.ב./השיב | term | ספר_ג'יבלי_אלמוקבאלא#OVQV | תשיב אל שרש |
| transformation between kinds/to transform between kinds | ש.ו.ב./השיב | term | ספר_ג'יבלי_אלמוקבאלא#Pvy2 | תשיב המספר למין השרש |
| transformation between kinds/to transform between kinds | ש.ו.ב./השיב | term | ספר_ג'יבלי_אלמוקבאלא#QYBE | השיבונו ל |
| transformation between kinds/to transform between kinds | ש.ו.ב./הושב | term | ספר_ג'יבלי_אלמוקבאלא#k75H | יושב |
| transformation between kinds/to transform between kinds | ש.ו.ב./השיב | term | ספר_ג'יבלי_אלמוקבאלא#f1HX | השיבות ה |
| transformation between kinds/to transform between kinds | ש.ו.ב./השיב | term | ספר_ג'יבלי_אלמוקבאלא#ET7N | תשיב המספר אל שרש |
| transformation between kinds/to transform between kinds | ש.ו.ב./השיב | term | ספר_ג'יבלי_אלמוקבאלא#qStG | השב ה |
| transformation between kinds/to transform between kinds | ש.ו.ב./השיב | term | ספר_ג'יבלי_אלמוקבאלא#uLzJ | בהשיב |
| transformation between kinds/to transform between kinds | ש.ו.ב./השבה | term | ספר_ג'יבלי_אלמוקבאלא#G4lq | השבה |
| algebraic operation/transformation between kinds | ש.ו.ב./השבה | term | ספר_ג'יבלי_אלמוקבאלא#R41D | השבת ה |
| equation/unsolvable equation | ט.ע.י./מוטעה | term | חשבון_השטחים#2srD | מוטעה |
| equation/unsolvable equation | ש.ב.ש./משובש | term | Anonymous#Jf2K | משובשת |
| equation/unsolvable equation | כ.ז.ב./כוזב | term | תחבולות_המספר#PMZL | כוזבת |
| algebraic species/x | ד.ב.ר./דבר | term | ספר_ג'יבלי_אלמוקבאלא#vEta | דבר |
| algebraic species/x | ש.ר.ש./שרש | term | ספר_ג'יבלי_אלמוקבאלא#oap6 | שורש |
| algebraic species/x | ע.ל.מ./נעלם | term | ספר_האלזיברא#kpod | דבר נעלם |
| algebraic species/x | ש.ר.ש./שרש | term | אגרת_המספר#3AcE | שרש |
| algebraic species/x | ע.ל.מ./נעלם | term | ספר_האלזיברא#gMmp | המספר הנעלם |
| algebraic species/x | ד.ב.ר./דבר | term | חשבון_השטחים#T2wp | דבר |
| algebraic species/x | ש.ר.ש./שרש | term | ספר_ג'יבלי_אלמוקבאלא#FM7v | שרש הצינסו |
| algebraic species/x | ד.ב.ר./דבר | term | אגרת_המספר#Cjip | דברים |
| algebraic species/x | צלע | term | ספר_ג'יבלי_אלמוקבאלא#03nK | צלע הצינסו |
| algebraic species/x | ש.ר.ש./שרש | term | תחבולות_המספר#Eu3f | שרש המרובע |
| algebraic species/x | ש.ר.ש./שרש | term | אגרת_המספר#2OZO | שרשים |
| algebraic species/x | ד.ב.ר./דבר | definition | אגרת_המספר#MRiG | things are roots. הדברים הם השרשים |
| algebraic species/x | ש.ר.ש./שרש | term | תחבולות_המספר#Dwqh | שרשים |
| algebraic species/x | ש.ר.ש./שרש | term | תחבולות_המספר#EURV | שרש מרובע |
| algebraic species/x | ש.ר.ש./שרש | definition | אגרת_המספר#XmEg | the roots are the roots of the squares. והשרשים הם שרשי המרובעים |
| algebraic species/x | ד.ב.ר./דבר | term | אגרת_המספר#IW0C | דבר |
| algebraic species/x | term | חשבון_השטחים#5pEs | ראדיש | |
| algebraic species/x | ד.ב.ר./דבר | term | חשבון_השטחים#HZEr | דברים |
| algebraic species/x | ד.ב.ר./דבר | term | תחבולות_המספר#7shv | דבר |
| algebraic species/x | ד.ב.ר./דבר | term | ספר_החשבון_לאל_חצאר#RZ9T | דברים |
| algebraic species/x | ד.ב.ר./דבר | term | תחבולות_המספר#EDBb | דברים |
| algebraic species/x | ד.ב.ר./דבר | term | ספר_האלזיברא#xvqw | דבר |
| algebraic species/x | definition | חשבון_השטחים#RMfA | The Root is anything that is multiplied by itself - as the one and upwards as well as its fractions and its fractions of fractions endlessly.
והשרש הוא כל דבר שיוכה [ש{{#annot:term|358,1587|On98}}יתרבה{{#annotend:On98}}]marg. על עצמו | |
| algebraic species/x | definition | תחבולות_המספר#YNih | The root is the number that is multiplied by itself - as if you say: one by one, two by two, and so on endlessly - and also the fractions of the one, when they are multiplied by themselves, as a half by a half, a third by a third and the fractions of its fractions and so on endlessly. השרש הוא המנין שהוא מוכה על עצמו כאלו תאמר אחד על אחד ושנים על שנים וכן לאין תכלית וכמו כן שברי האחד כאשר הוכו על עצמם כמו חצי על חצי ושלישית על שלישית וכן שברי שבריו עד אין סוף | |
| algebraic species/x | ד.ב.ר./דבר | term | ספר_החשבון_לאל_חצאר#zuis | דבר |
| algebraic species/x | ש.ר.ש./שרש | term | חשבון_השטחים#SFi3 | שרשים |
| algebraic species/x | קוסא | term | ספר_האלזיברא#GJ6c | קוֹסָא |
| algebraic species/x² | ר.ב.ע./מרובע | term | תחבולות_המספר#nx6j | מרובעים |
| algebraic species/x² | definition | תחבולות_המספר#CftH | The square is what is produced from the multiplication of the root by itself - be it an integer or a fraction. המרובע הוא המתקבץ מהכאת השרש על עצמו {{#annot: term | #integer, #שלם | Kd1b}}שלם{{#annotend:Kd1b}} יהיה או {{#annot: term | #fraction, #נשבר | jSnE}}נשבר{{#annotend:jSnE}} | |
| algebraic species/x² | אלגו | term | חשבון_השטחים#BEDv | אלגו |
| algebraic species/x² | definition | חשבון_השטחים#hXNL | The square is what is produced from the multiplication of the root by itself - be it an integer or a fraction. וה{{#annot:term|687,1304|lQK4}}אלגו{{#annotend:lQK4}} הוא המתקבץ מ{{#annot:term|156,2068|LBvR}}התרבות{{#annotend:LBvR}} זה השרש על עצמו {{#annot:term|20,1268|DXqj}}שלם{{#annotend:DXqj}} יהיה או {{#annot:term|15,1438|Xlsp}}נשבר{{#annotend:Xlsp}} | |
| algebraic species/x² | ר.ב.ע./מרובע | term | חשבון_השטחים#e4zw | מרובעי' |
| algebraic species/x² | צינסו | term | ספר_ג'יבלי_אלמוקבאלא#TF4O | צינסו |
| algebraic species/x² | צינסו | term | ספר_ג'יבלי_אלמוקבאלא#uHk9 | צינסי |
| algebraic species/x² | מ.מ.נ./ממון | term | אגרת_המספר#aGa5 | ממונות |
| algebraic species/x² | צינסו | term | ספר_האלזיברא#c1bw | צֵינְסו |
| algebraic species/x² | מ.מ.נ./ממון | definition | אגרת_המספר#zZE7 | squares are the product of the root multiplied by itself. והממונות מה שיתקבץ מן השרש מוכה בעצמו |
| algebraic species/x² | מ.מ.נ./ממון | term | אגרת_המספר#jMz0 | ממון |
| algebraic species/x² | ר.ב.ע./מרובע | term | ספר_האלזיברא#vYMJ | מרובע |
| algebraic species/x² | ר.ב.ע./מרובע | term | ספר_האלזיברא#xdn2 | מרובע |
| algebraic species/x² | אלגו | term | חשבון_השטחים#lQK4 | אלגו |
| algebraic species/x³ | קוביקא | term | חשבון_השטחים#Ou2h | קוביקש |
| algebraic species/x³ | ע.ק.ב./מעוקב | term | ספר_ג'יבלי_אלמוקבאלא#ag26 | מעוקבים |
| algebraic species/x³ | קוביקא | term | חשבון_השטחים#vsRc | קוביקא |
| algebraic species/x³ | ע.ק.ב./מעוקב | term | חשבון_השטחים#PZlk | מעוקב |
| algebraic species/x³ | ע.ק.ב./מעוקב | term | ספר_האלזיברא#V0Fq | מספר המעקב |
| algebraic species/x³ | ע.ק.ב./מעוקב | term | חשבון_השטחים#ykLi | מעוקבים |
| algebraic species/x³ | ע.ק.ב./מעוקב | term | אגרת_המספר#66vb | מעוקבים |
| algebraic species/x³ | קובו | term | ספר_האלזיברא#wegk | קוּבוּ |
| algebraic species/x³ | ע.ק.ב./מעוקב | term | ספר_ג'יבלי_אלמוקבאלא#4mEv | מעוקב |
| algebraic species/x³ | ע.ק.ב./מעוקב | term | אגרת_המספר#lFty | מעוקב |