Difference between revisions of "Mathematical formula"
From mispar
| Line 4: | Line 4: | ||
<math>\scriptstyle\left(3n+1\right)^2=\left[\left[10\sdot\left[\frac{1}{3}\sdot\left(3n\right)\right]^2\right]-\left[\frac{1}{3}\sdot\left(3n\right)\right]^2\right]+3n+\left(3n+1\right)</math>]] | <math>\scriptstyle\left(3n+1\right)^2=\left[\left[10\sdot\left[\frac{1}{3}\sdot\left(3n\right)\right]^2\right]-\left[\frac{1}{3}\sdot\left(3n\right)\right]^2\right]+3n+\left(3n+1\right)</math>]] | ||
| + | <div class="mw-collapsible mw-collapsed"><math>\scriptstyle bx=\sqrt[3]{c}</math><div class="mw-collapsible-content"> | ||
{{#annotask: | {{#annotask: | ||
[[category: #linear equation]] | [[category: #linear equation]] | ||
[[comment: bx=³√c]] | [[comment: bx=³√c]] | ||
| − | }} | + | }}</div></div> |
| + | <br> | ||
| + | <div class="mw-collapsible mw-collapsed"><math>\scriptstyle c=\sqrt[3]{bx}</math><div class="mw-collapsible-content"> | ||
{{#annotask: | {{#annotask: | ||
[[category: #linear equation]] | [[category: #linear equation]] | ||
[[comment: c=³√bx]] | [[comment: c=³√bx]] | ||
| − | }} | + | }}</div></div> |
| + | <br> | ||
| + | <div class="mw-collapsible mw-collapsed"><math>\scriptstyle ax^2=\sqrt[3]{c}</math><div class="mw-collapsible-content"> | ||
{{#annotask: | {{#annotask: | ||
[[category: #quadratic equation]] | [[category: #quadratic equation]] | ||
[[comment: ax²=³√c]] | [[comment: ax²=³√c]] | ||
| − | }} | + | }}</div></div> |
| + | <br> | ||
| + | <div class="mw-collapsible mw-collapsed"><math>\scriptstyle c=\sqrt[3]{ax^2}</math><div class="mw-collapsible-content"> | ||
{{#annotask: | {{#annotask: | ||
[[category: #quadratic equation]] | [[category: #quadratic equation]] | ||
[[comment: c=³√ax²]] | [[comment: c=³√ax²]] | ||
| − | }} | + | }}</div></div> |
| + | <br> | ||
| + | <div class="mw-collapsible mw-collapsed"><math>\scriptstyle ax^3=\sqrt[3]{c}</math><div class="mw-collapsible-content"> | ||
{{#annotask: | {{#annotask: | ||
[[category: #cubic equation]] | [[category: #cubic equation]] | ||
[[comment: ax³=³√c]] | [[comment: ax³=³√c]] | ||
| − | }} | + | }}</div></div> |
| − | + | <br> | |
| + | <div class="mw-collapsible mw-collapsed"><math>\scriptstyle4\sdot\left(x^2+8\right)=x^4</math><div class="mw-collapsible-content"> | ||
{{#annotask: | {{#annotask: | ||
[[category: #biquadratic equation]] | [[category: #biquadratic equation]] | ||
[[comment: 4(x²+8)=x⁴]] | [[comment: 4(x²+8)=x⁴]] | ||
| − | }} | + | }}</div></div> |
Revision as of 08:33, 7 April 2019
![\scriptstyle\left(3n+1\right)^2=\left[\left[10\sdot\left[\frac{1}{3}\sdot\left(3n\right)\right]^2\right]-\left[\frac{1}{3}\sdot\left(3n\right)\right]^2\right]+3n+\left(3n+1\right)](/mediawiki/images/math/2/a/f/2af90eb131ece9e62879b03d8922a419.png)
![\scriptstyle bx=\sqrt[3]{c}](/mediawiki/images/math/4/e/c/4eced3ba1c8f51bf47cf911659b6e201.png)
| Category | Comment | Link | Annotated text |
|---|---|---|---|
| equation/linear equation | bx=³√c | ספר_ג'יבלי_אלמוקבאלא#NHZd | When things are equal to a cube root of the numbers:
:
כאשר הדברי' יהיו שוי' אל שרש מעוק' ממספרי' |
![\scriptstyle c=\sqrt[3]{bx}](/mediawiki/images/math/5/b/4/5b40a2184fb3c6324ff5fe2eedb9da0b.png)
| Category | Comment | Link | Annotated text |
|---|---|---|---|
| equation/linear equation | c=³√bx | ספר_ג'יבלי_אלמוקבאלא#jGFm | When numbers are equal to a cube root of a thing:
:
כאשר המספרי' יהיו שוים אל שרש מעו' מדבר |
![\scriptstyle ax^2=\sqrt[3]{c}](/mediawiki/images/math/f/c/0/fc0033e1c190540235d4afbc47b31b11.png)
| Category | Comment | Link | Annotated text |
|---|---|---|---|
| equation/quadratic equation | ax²=³√c | ספר_ג'יבלי_אלמוקבאלא#rGD5 | When squares are equal to a cube root of the numbers:
:
כאשר הצינסי יהיו שוים אל שרשי' מעו' ממספרי' |
![\scriptstyle c=\sqrt[3]{ax^2}](/mediawiki/images/math/f/7/6/f7617468fb35452327a7d2d5678e45f0.png)
| Category | Comment | Link | Annotated text |
|---|---|---|---|
| equation/quadratic equation | c=³√ax² | ספר_ג'יבלי_אלמוקבאלא#zK2y | When numbers are equal to a cube root of squares:
:
כאשר המספרי' יהיו שוים אל שרשי' מעו' מצינסי |
![\scriptstyle ax^3=\sqrt[3]{c}](/mediawiki/images/math/a/6/4/a64e6eaeaf2d5f64610f741998d43d86.png)
| Category | Comment | Link | Annotated text |
|---|---|---|---|
| equation/cubic equation | ax³=³√c | ספר_ג'יבלי_אלמוקבאלא#eOI5 | It is when cubes are equal to a cube root of the numbers:
:
וזהו כאשר המעוקבי' יהיו שוים אל שרש מעו' ממספרי' |
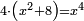
| Category | Comment | Link | Annotated text |
|---|---|---|---|
| quartic equation/biquadratic equation | 4(x²+8)=x⁴ | אגרת_המספר#q2Fw | 6)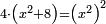 
הששית ממון הוספת עליו ח' זוזים והכית המקובץ בארבעה והיה היוצא הכאת הממון בעצמו |
| quartic equation/biquadratic equation | 4(x²+8)=x⁴ | תחבולות_המספר#G1Mq | [6] He said: the six problem is as if you are told: we add to a certain square [eight] dirham, then multiply the sum by four dirham and the result is the same as the product of the square [by itself].
:
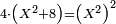אמ' והשאלה הששית כמו אם יאמרו לך הוספנו על |
| quartic equation/biquadratic equation | 4(x²+8)=x⁴ | חשבון_השטחים#ZxMx |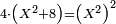
אלגו תוסיף עליו שמנה אדרהם ותכה {{#annot:term|388,1217|vQSL}}המקובץ{{#annotend:vQSL}} על ארבעה אדרהם והיה כמו האלגו על עצמו |
