Difference between revisions of "Mathematical formula"
From mispar
| Line 3: | Line 3: | ||
[[(3n+1)²=((10·(⅓·3n)²)-(⅓·3n)²)+3n+(3n+1)| | [[(3n+1)²=((10·(⅓·3n)²)-(⅓·3n)²)+3n+(3n+1)| | ||
<math>\scriptstyle\left(3n+1\right)^2=\left[\left[10\sdot\left[\frac{1}{3}\sdot\left(3n\right)\right]^2\right]-\left[\frac{1}{3}\sdot\left(3n\right)\right]^2\right]+3n+\left(3n+1\right)</math>]] | <math>\scriptstyle\left(3n+1\right)^2=\left[\left[10\sdot\left[\frac{1}{3}\sdot\left(3n\right)\right]^2\right]-\left[\frac{1}{3}\sdot\left(3n\right)\right]^2\right]+3n+\left(3n+1\right)</math>]] | ||
| + | |||
| + | |||
| + | == Linear Equation == | ||
<div class="mw-collapsible mw-collapsed"><math>\scriptstyle bx=\sqrt[3]{c}</math><div class="mw-collapsible-content"> | <div class="mw-collapsible mw-collapsed"><math>\scriptstyle bx=\sqrt[3]{c}</math><div class="mw-collapsible-content"> | ||
| Line 16: | Line 19: | ||
}}</div></div> | }}</div></div> | ||
<br> | <br> | ||
| + | |||
| + | |||
| + | == Quadratic Equation == | ||
| + | |||
<div class="mw-collapsible mw-collapsed"><math>\scriptstyle ax^2=\sqrt[3]{c}</math><div class="mw-collapsible-content"> | <div class="mw-collapsible mw-collapsed"><math>\scriptstyle ax^2=\sqrt[3]{c}</math><div class="mw-collapsible-content"> | ||
{{#annotask: | {{#annotask: | ||
| Line 28: | Line 35: | ||
}}</div></div> | }}</div></div> | ||
<br> | <br> | ||
| + | |||
| + | |||
| + | == Cubic Equation == | ||
| + | |||
<div class="mw-collapsible mw-collapsed"><math>\scriptstyle ax^3=\sqrt[3]{c}</math><div class="mw-collapsible-content"> | <div class="mw-collapsible mw-collapsed"><math>\scriptstyle ax^3=\sqrt[3]{c}</math><div class="mw-collapsible-content"> | ||
{{#annotask: | {{#annotask: | ||
| Line 34: | Line 45: | ||
}}</div></div> | }}</div></div> | ||
<br> | <br> | ||
| + | |||
| + | == Biquadratic Equation == | ||
| + | |||
<div class="mw-collapsible mw-collapsed"><math>\scriptstyle4\sdot\left(x^2+8\right)=x^4</math><div class="mw-collapsible-content"> | <div class="mw-collapsible mw-collapsed"><math>\scriptstyle4\sdot\left(x^2+8\right)=x^4</math><div class="mw-collapsible-content"> | ||
{{#annotask: | {{#annotask: | ||
Revision as of 04:42, 9 April 2019
Linear Equation
[Expand]![\scriptstyle bx=\sqrt[3]{c}](/mediawiki/images/math/4/e/c/4eced3ba1c8f51bf47cf911659b6e201.png)
![\scriptstyle bx=\sqrt[3]{c}](/mediawiki/images/math/4/e/c/4eced3ba1c8f51bf47cf911659b6e201.png)
[Expand]![\scriptstyle c=\sqrt[3]{bx}](/mediawiki/images/math/5/b/4/5b40a2184fb3c6324ff5fe2eedb9da0b.png)
![\scriptstyle c=\sqrt[3]{bx}](/mediawiki/images/math/5/b/4/5b40a2184fb3c6324ff5fe2eedb9da0b.png)
Quadratic Equation
[Expand]![\scriptstyle ax^2=\sqrt[3]{c}](/mediawiki/images/math/f/c/0/fc0033e1c190540235d4afbc47b31b11.png)
![\scriptstyle ax^2=\sqrt[3]{c}](/mediawiki/images/math/f/c/0/fc0033e1c190540235d4afbc47b31b11.png)
[Expand]![\scriptstyle c=\sqrt[3]{ax^2}](/mediawiki/images/math/f/7/6/f7617468fb35452327a7d2d5678e45f0.png)
![\scriptstyle c=\sqrt[3]{ax^2}](/mediawiki/images/math/f/7/6/f7617468fb35452327a7d2d5678e45f0.png)
Cubic Equation
[Expand]![\scriptstyle ax^3=\sqrt[3]{c}](/mediawiki/images/math/a/6/4/a64e6eaeaf2d5f64610f741998d43d86.png)
![\scriptstyle ax^3=\sqrt[3]{c}](/mediawiki/images/math/a/6/4/a64e6eaeaf2d5f64610f741998d43d86.png)
Biquadratic Equation
[Expand]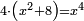
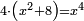
![\scriptstyle\left(3n+1\right)^2=\left[\left[10\sdot\left[\frac{1}{3}\sdot\left(3n\right)\right]^2\right]-\left[\frac{1}{3}\sdot\left(3n\right)\right]^2\right]+3n+\left(3n+1\right)](/mediawiki/images/math/2/a/f/2af90eb131ece9e62879b03d8922a419.png)
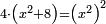 הששית ממון הוספת עליו ח' זוזים והכית המקובץ בארבעה והיה היוצא הכאת הממון בעצמו
הששית ממון הוספת עליו ח' זוזים והכית המקובץ בארבעה והיה היוצא הכאת הממון בעצמו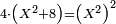 אמ' והשאלה הששית כמו אם יאמרו לך הוספנו על
אמ' והשאלה הששית כמו אם יאמרו לך הוספנו על