Difference between revisions of "Search Page"

Latest revision as of 12:36, 6 September 2019

Category	Category	Comment	Link	Annotated text
quadratic equation/canonical equation	ח.ל.ק./חלק	term	חשבון_השטחים#rsWG	הששה חלקים
quadratic equation/canonical equation		term	חשבון_השטחים#hEUg	הששה בקשות
canonical equation/compound canonical equation	ח.ב.ר./מחובר	term	חשבון_השטחים#y7Pt	מחוברים
canonical equation/compound canonical equation		definition	חשבון_השטחים#FjYV	ושלשה {{#annot: term \| #compound canonical equation, #מחובר \| y7Pt}}מחוברים{{#annotend:y7Pt}} והם אלגוש ושרשים ישוו מספרים ואלגוש ומספרים ישוו שרשים ושרשים ומספרים ישוו אלגוש
equation/quadratic equation		(x²-⅓x²)·3√x²=x²	חשבון_השטחים#ak8r	[14] If you are told: we subtract from a square its third, then multiply what remains by three roots of the original square and the result is the original square. :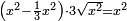 $\scriptstyle\left(x^2-\frac{1}{3}x^2\right)\sdot3\sqrt{x^2}=x^2$ ואם יאמרו לך גרענו מן האלגו שלישיתו והכינו הנשאר על שלשה שרשים מהאלגו הראשון ושב האלגו הראשון
equation/quadratic equation		[x²-(⅓x²+2)]²=x²+24	חשבון_השטחים#lT08	[12] If you are told: a square, you subtract its third and two dirham from it, then multiply what remains by itself and the result is the square plus 24 dirham. : $\scriptstyle\left[x^2-\left(\frac{1}{3}x^2+2\right)\right]^2=x^2+24$ ואם יאמרו לך אלגו גרעת ממנו שלישיתו ושני אדרהמיש ומה שנשאר הכית אותו על עצמו ושב האלגו וכ"ד אדרהמיש יותר
equation/quadratic equation		[√(6x²)·√(5x²)]+10x²+20=(x²)²	חשבון_השטחים#stdc	[34] If you are told: a square, multiply a root of its six times by a root of its five times, then add ten times the square plus twenty dirham to the product and it is the same as [the product of] the square by itself. :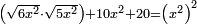 $\scriptstyle\left(\sqrt{6x^2}\sdot\sqrt{5x^2}\right)+10x^2+20=\left(x^2\right)^2$ ואם יאמרו לך אלגו תרבה שרש מששה דמיוניו בשרש חמשה דמיוניו והוספת על מה שעלה מההכאה עשרה דמיוני האלגו ועשרים דרהמי יותר ויהיה כמו האלגו על עצמו
equation/quadratic equation		[√(8x²)·√(3x²)]+20=(x²)²	חשבון_השטחים#HcHI	[33] If you are told: a square, multiply a root of eight times the square by a root of three times the square, then add twenty dirham to the result and it is the same as [the product of] the square by itself. :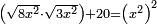 $\scriptstyle\left(\sqrt{8x^2}\sdot\sqrt{3x^2}\right)+20=\left(x^2\right)^2$ ואם יאמרו לך אלגו תרבה שרש משמנה דמיוני האלגו בשרש משלשה דמיוני האלגו והוספת על העולה עשרים דרהמי ויהיה כמו האלגו על עצמו
equation/quadratic equation		(x²-⅓x²)·3√(x²-⅓x²)=x²	חשבון_השטחים#lFM5	[15] If you are told: a square, we subtract its third from it, then multiply what remains by three roots of what remains from the square and the result is the square. : $\scriptstyle\left(x^2-\frac{1}{3}x^2\right)\sdot3\sqrt{x^2-\frac{1}{3}x^2}=x^2$ ואם יאמרו לך אלגו גרענו ממנו שלישיתו והכינו הנשאר על שלשה שרשים מהנשאר מהאלגו וישוב האלגו
equation/quadratic equation		a²+b²=c², ac=b², ab=10	חשבון_השטחים#gGKe	If you are told: three unequal squares, if you multiply the smaller by itself and the mean by itself; they are as the greater by itself. If you multiply the smaller by the greater it is as the mean by itself. If you multiply the smaller by the mean it is ten dirham. :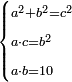 $\scriptstyle\begin{cases}\scriptstyle a^2+b^2=c^2\\\scriptstyle a\sdot c=b^2\\\scriptstyle a\sdot b=10\end{cases}$ ואם יאמרו לך שלשה אלגוש בלתי שוים אם תכה הקטן בעצמו והאמצעי בעצמו יהיו כמו הגדול בעצמו ואם תכה הקטון בגדול יהיה כמו האמצעי בעצמו ואם תכה הקטן באמצעי יהיה עשרה דרהמי
equation/quadratic equation		b²=3a², (a²+√a²)·(b²+√b²)=10b²	חשבון_השטחים#nHFm	If you are told: two squares - one is three times the other; you add to each of them its root, then multiply the one by the other; it is ten times the greater square. : $\scriptstyle\begin{cases}\scriptstyle b^2=3a^2\\\scriptstyle\left(a^2+\sqrt{a^2}\right)\sdot\left(b^2+\sqrt{b^2}\right)=10b^2\end{cases}$ ואם יאמרו שני אלגוש והאחד שלשה דמיוני האחר הוספת על כל אחד מהם שרשו שלו והכית האחד באחר והיה עשרה דמיוני האלגו הגדול
equation/quadratic equation		[x²+√(½x²)]²=4x²	חשבון_השטחים#Fvgh	If you are told: a square, you add to it a root of its half, then multiply the result by itself; it becomes four times the square. :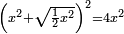 $\scriptstyle\left(x^2+\sqrt{\frac{1}{2}x^2}\right)^2=4x^2$ ואם יאמרו לך אלגו הוספת עליו שרש חציו והכית העולה בעצמו ויהיה ארבעה דמיוני האלגו
equation/quadratic equation		[x²+√(x²)+√(½x²)]²=5x²	חשבון_השטחים#omoo	If you are told: a square, add to it its root and a root of its half, then multiply the result by itself; it is five times the square. :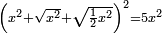 $\scriptstyle\left(x^2+\sqrt{x^2}+\sqrt{\frac{1}{2}x^2}\right)^2=5x^2$ ואם יאמרו לך אלגו תוסיף עליו שרשו ושרש חציו ותכה המקובץ על עצמו ויהיה חמשה דמיוני האלגו
equation/quadratic equation		x²+4√(x²)+√(½x²)+√(⅓x²)=10	חשבון_השטחים#b04G	If you are told: a square, add to it its four roots plus a root of its half and a root of its third; it is ten dirham - how much is the square? : $\scriptstyle x^2+4\sqrt{x^2}+\sqrt{\frac{1}{2}x^2}+\sqrt{\frac{1}{3}x^2}=10$ ואם יאמר לך אלגו תוסיף עליו ארבעה שרשיו ושרש חציו ושרש שלישיתו ויהיה עשרה דרהמי כמה הוא האלגו
equation/quadratic equation		2√(x²)+√(½x²)+√(⅓x²)=20	חשבון_השטחים#wOZV	If one says: a square whose two roots plus a root of its half and a root of its third are twenty dirham - how much is the square? :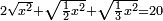 $\scriptstyle2\sqrt{x^2}+\sqrt{\frac{1}{2}x^2}+\sqrt{\frac{1}{3}x^2}=20$ ואם יאמר אלגו אשר שני שרשיו ושרש חציו ושרש שלישיתו עשרים דרהמי כמה הוא האלגו
equation/quadratic equation		[x²-(2√x²+10)]²=8x²	חשבון_השטחים#aPQX	If you are told: a square, subtract its two roots and ten dirham from it, then multiply what remains by itself; it becomes eight times the square. : $\scriptstyle\left[x^2-\left(2\sqrt{x^2}+10\right)\right]^2=8x^2$ ואם יאמרו לך אלגו תגרע ממנו שני שרשיו ועשרה דרהמי ותכה מה שישאר בעצמו ויהיה שמנה דמיוני האלגו
equation/quadratic equation		[√(x²·2x²)+2]·x²=30	חשבון_השטחים#uwLQ	[40] If you are told: a square, you multiply it by its two times, extract the root of the product, add two dirham to it, then multiply the total by that square and the result is thirty dirham. :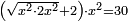 $\scriptstyle\left(\sqrt{x^2\sdot2x^2}+2\right)\sdot x^2=30$ ואם יאמרו לך אלגו תכהו על {{#annot:term\|387\|piMO}}שני דמיוניו{{#annotend:piMO}} ותקח שרש העולה ותוסיף עליו שני דרהמי ותכה הכל באלגו ההוא ויהיה שלשים דרהמי
equation/quadratic equation		(x²+10)·√5=(x²)²	חשבון_השטחים#hePu	[38] If you are told: a square, you add to it ten dirham, then multiply the sum by a root of five and the result is the same as the product of the square by itself. :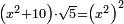 $\scriptstyle\left(x^2+10\right)\sdot\sqrt{5}=\left(x^2\right)^2$ ואם יאמרו לך אלגו הוספת עליו עשרה דרהמי והכית המקובץ בשרש חמשה ויעלה כמו הכאת האלגו על עצמו
equation/quadratic equation		3√x²+4√(x²-3√x²)=x²+4	חשבון_השטחים#SNBo	[17] If you are told: a square, its three roots and four roots of what remains from the square are the same as the square plus four dirham. :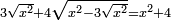 $\scriptstyle3\sqrt{x^2}+4\sqrt{x^2-3\sqrt{x^2}}=x^2+4$ ואם יאמרו לך אלגו שלשת שרשיו וארבעה שרשים ממה שנשאר מהאלגו יהיה כמו האלגו וארבעה דרהמי' יותר
equation/quadratic equation		3√x²+2√(x²-3√x²)=x²	חשבון_השטחים#rq9x	[16] If you are told: a square, its three roots and two roots of what remains are equal to the square. :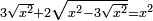 $\scriptstyle3\sqrt{x^2}+2\sqrt{x^2-3\sqrt{x^2}}=x^2$ ואם יאמרו לך אלגו שלשת שרשיו ושני שרשי הנשאר ישוו האלגו
equation/quadratic equation		[x²+√(x²)+√(½x²)]²=20	חשבון_השטחים#OX0E	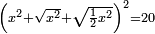$\scriptstyle\left(x^2+\sqrt{x^2}+\sqrt{\frac{1}{2}x^2}\right)^2=20$ ואם יאמרו לך אלגו הוסף עליו שרשו ושרש חציו ותכה המקובץ בעצמו ויהיה עשרים דרהמי
equation/quadratic equation		2√(x²)+√(½x²)+√(⅓x²)=x²	חשבון_השטחים#nPTX	If you are told: a square whose two roots plus a root of half the square and a root of its third are equal to the square - how much is the square? : $\scriptstyle2\sqrt{x^2}+\sqrt{\frac{1}{2}x^2}+\sqrt{\frac{1}{3}x^2}=x^2$ ואם יאמרו לך אלגו אשר שני שרשיו ושרש חצי האלגו ושרש שלישיתו ישוו האלגו כמה הוא האלגו
equation/quadratic equation		√x²+√(√x²)+√(2√x²)+5√x²=10	חשבון_השטחים#rWIe	If you are told: a square whose root and the root of its root, plus the root of its two roots, plus the root of five times the square are ten dirham. :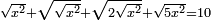 $\scriptstyle\sqrt{x^2}+\sqrt{\sqrt{x^2}}+\sqrt{2\sqrt{x^2}}+\sqrt{5x^2}=10$ ואם יאמרו לך אלגו אשר שרשו ו{{#annot:term\|2634\|dE2e}}שרש שרשו{{#annotend:dE2e}} ושרש שני שרשיו ושרש חמשה דמיוני האלגו יהיה עשרה דרהמי
equation/quadratic equation		x²·(x²+√10)=9x²	חשבון_השטחים#XpMD	[32] If you are told: a square, multiply it by itself plus a root of ten dirham and it becomes nine times the square. :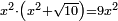 $\scriptstyle x^2\sdot\left(x^2+\sqrt{10}\right)=9x^2$ ואם יאמרו לך אלגו תכהו על עצמו ושרש אחד מעשרה דרהמי ויהיה תשעה דמיוני האלגו
equation/quadratic equation		3√x²+4√(x²-3√x²)=20	חשבון_השטחים#tZ8i	[13] If you are told: three roots of the square plus four roots of what remains from the square are twenty dirham. :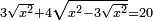 $\scriptstyle3\sqrt{x^2}+4\sqrt{x^2-3\sqrt{x^2}}=20$ ואם יאמרו לך שלשה שרשים מהאלגו וארבעה שרשים ממה שנשאר מהאלגו יהיה עשרים דרהמי‫'
equation/quadratic equation		(√10·x²)/(2√3)=x²-10	חשבון_השטחים#8OnP	If you are told: a square, multiply it by the root of ten, then divide the product by two plus the root of three; the quotient is the same as the square minus ten. :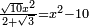 $\scriptstyle\frac{\sqrt{10}x^2}{2+\sqrt{3}}=x^2-10$ ואם יאמרו לך אלגו תכהו בשרש עשרה ותחלק מה שיעלה על שנים ושרש שלשה ויעלה לאחד כל כך כמו שהוא האלגו פחות עשרה
equation/quadratic equation		[√(½x²)+3]·[√(⅓x²)+2]=20	חשבון_השטחים#Y7kO	If you are told: a square, add three dirham to a root of its half, and two dirham to a root of its third, then multiply one [sum] by the other; it is twenty dirham. :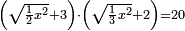 $\scriptstyle\left(\sqrt{\frac{1}{2}x^2}+3\right)\sdot\left(\sqrt{\frac{1}{3}x^2}+2\right)=20$ ואם יאמרו לך אלגו תוסיף על שרש חציו שלשה דרהמי ועל שרש שלישיתו שני דרהמי ותכה האחד באחר ויהיה עשרים דרהמי
equation/quadratic equation		[x²+√(3x²)]·√(2x²)=4x²	חשבון_השטחים#lk6C	If you are told: a square, add to it a root of three times of it, then multiply the sum by a root of [twice] the square; it becomes four times the square. :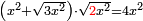 $\scriptstyle\left(x^2+\sqrt{3x^2}\right)\sdot\sqrt{{\color{red}{2}}x^2}=4x^2$ ואם יאמרו לך אלגו תוסיף עליו שרש שלשה דמיוניו ותכה המקובץ בשרש האלגו יהיה ארבעה דמיוני האלגו
equation/quadratic equation		(x²+7)·√(3x²)=10x²	חשבון_השטחים#dAZJ	If you are told: a square, add to it seven dirham, then multiply the sum by a root of three times the square; it becomes ten times the square. :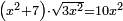 $\scriptstyle\left(x^2+7\right)\sdot\sqrt{3x^2}=10x^2$ ואם יאמרו לך אלגו תוסיף עליו שבעה דרהמי ותכה המקובץ בשרש שלשה דמיוני האלגו ויהיה עשרה דמיוני האלגו
compound canonical equation/roots and numbers equal squares		bx+c=ax²	תחבולות_המספר#So10	ושרשי' ומספרים שישוו למרובעים
compound canonical equation/roots and numbers equal squares		3x+4=x²	תחבולות_המספר#VKnl	He said: roots and numbers that are equal to a square is as saying three roots and four dirham are equal to a square. :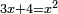 $\scriptstyle3x+4=x^2$ אמ' שרשים ומספרים שישוו למרובע הוא כמו שיאמ' שלשה שרשים וארבעה דרהמי יהיו שוים למרובע
compound canonical equation/roots and numbers equal squares		3x+4=x²	חשבון_השטחים#qrpb	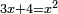$\scriptstyle3x+4=x^2$ והשרשים ומספרים שישוו אלגוש כמו אם יאמר שלשה שרשים וארבעה מספרים ישוו אלגו
compound canonical equation/roots and numbers equal squares		bx+c=ax²	חשבון_השטחים#VBed	ושרשים ומספרי' ישוו למרובעים
simple canonical equation/roots equal numbers		½x=10	תחבולות_המספר#e4m4	If one says: half a root is equal to ten. :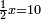 $\scriptstyle\frac{1}{2}x=10$ ואם אמ' ~~חמשה ושלשים~~ חצי שרש ישוה לעשרה
simple canonical equation/roots equal numbers		bx=c	תחבולות_המספר#qsBh	ושרשים שישוו למספרים
simple canonical equation/roots equal numbers		5x=30	חשבון_השטחים#1YGh	Also if you say: five roots are equal to thirty. :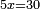 $\scriptstyle5x=30$ וכמו אם תאמר חמשה שרשים ישוו שלשים
simple canonical equation/roots equal numbers		x=4	חשבון_השטחים#wMvn	When the roots are equal to numbers, as if you say: the root is equal to four. :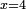 $\scriptstyle x=4$ והשרשים שישוו מספרים כמו שתאמר שרש ישוה ארבעה
simple canonical equation/roots equal numbers		bx=c	חשבון_השטחים#1nZH	ושרשים ישוו מספרים
simple canonical equation/roots equal numbers		x=4	תחבולות_המספר#PaMj	If you are told: the roots of the square are equal to four numbers. :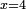 $\scriptstyle x=4$ כי אם יאמרו לך שרשי המרובע ישוו לארבע' מספרי‫'
simple canonical equation/roots equal numbers		½x=10	חשבון_השטחים#0Ojb	If you say: half a root is equal to ten. :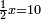 $\scriptstyle\frac{1}{2}x=10$ וכמו אם תאמר חצי שרש ישוה עשרה
simple canonical equation/roots equal numbers		5x=30	תחבולות_המספר#jHg9	As if one says: five roots are equal to thirty. :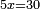 $\scriptstyle5x=30$ כמו אם אמ' חמשה שרשים ישוו לשלשים
canonical equation/simple canonical equation		definition	חשבון_השטחים#Ja1c	הנה השלשה מהם {{#annot: term \| #simple canonical equation, #נפרד \| o3Jf}}נפרדים{{#annotend:o3Jf}} והם אלגוש ישוו שרשים והאלגו ישוו מספרים ושרשים ישוו מספרים
canonical equation/simple canonical equation	פ.ר.ד./נפרד	term	חשבון_השטחים#o3Jf	נפרדים
canonical equation/simple canonical equation	פ.ש.ט./פשוט	term	תחבולות_המספר#Zvsu	הפשוטים
compound canonical equation/squares and numbers equal roots		ax²+c=bx	חשבון_השטחים#iECf	ומרובעים ומספרי' ישוו לשרשים
compound canonical equation/squares and numbers equal roots		x²+21=10x	חשבון_השטחים#yRRf	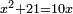$\scriptstyle x^2+21=10x$ והאלגוש והמספרים שישוו שרשים כאלו תאמר אלגוש ועשרים ואחד אדרהמיש ישוו עשרה שרשים
compound canonical equation/squares and numbers equal roots		ax²+c=bx	תחבולות_המספר#om8w	ומרובעי' ומספרי' שישוו לשרשים
compound canonical equation/squares and numbers equal roots		x²+25=10x	תחבולות_המספר#QWm3	Example: one says: a square plus twenty-five [dirham] are equal to ten roots of the square. :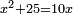 $\scriptstyle x^2+25=10x$ דמיון זה שיאמ' מרובע מה ועשרים וחמשה שרשים ישוו לעשרה שרשים מהמרובע
compound canonical equation/squares and numbers equal roots		x²+21=10x	תחבולות_המספר#u4EA	He said: squares and numbers that are equal to roots is as if you say: when you sum twenty-one dirham with a certain square, they are equal to ten roots of the square. :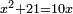 $\scriptstyle x^2+21=10x$ אמ' המרובעי' והמספרי' שישוו לשרשים הוא כאלו תאמ' כאשר תקבץ עם מרובע מה עשרים ואחד דרהמי יהיו שוים לעשרה משרשים מהמרובע
compound canonical equation/squares and numbers equal roots		x²+25=10x	חשבון_השטחים#5rN4	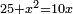$\scriptstyle25+x^2=10x$ ו{{#annot: term \| #to give an example, #משל \| ZpYe}}אמשיל זה{{#annotend:ZpYe}} בשאילה אחרת ואניחנה שכ"ה אדרהמיש ואלגוש ישוו עשרה שרשים
compound canonical equation/squares and roots equal numbers		x²+10x=39	חשבון_השטחים#BnUp	Squares and roots are equal to numbers, it is as if you say: a square and ten roots are equal to thirty-nine dirham. :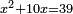 $\scriptstyle x^2+10x=39$ והאלגוש והשרשים שישוו מספרים ^הוא כאלו תאמר אלגו ועשרה שרשים ישוו תשעה ושלשים דרהמיש
compound canonical equation/squares and roots equal numbers		½x²+5x=28	תחבולות_המספר#FJRf	Likewise, if one asks: half a square plus its five roots are equal to 28 dirham. :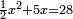 $\scriptstyle\frac{1}{2}x^2+5x=28$ וכמו כן אם שאל השואל חצי מרובע וחמשה משרשיו ישוו לכ"ח דרהמי
compound canonical equation/squares and roots equal numbers		3x²+15x=72	תחבולות_המספר#HV5b	Example: if one asks: three squares and 15 roots are equal to 72 dirham. :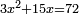 $\scriptstyle3x^2+15x=72$ דמיון זה ששאל שלשה מרובעי' וט"ו שרשי' ישוו לע"ב דרהמי
compound canonical equation/squares and roots equal numbers		x²+10x=39	תחבולות_המספר#oMPa	He said: when squares and roots are equal to numbers, it is as if you say: the sum of one square and ten of its roots together is equal to thirty-nine dirham. :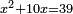 $\scriptstyle x^2+10x=39$ אמ' כאשר יהיו המרובעים והשרשים שוים למספרי' כאלו תאמ' המקובץ ממרובע מהאחד ועשרה משרשיו יחד ישוה לשלשים ותשעה דרהמי
compound canonical equation/squares and roots equal numbers		2x²+10x=48	חשבון_השטחים#cSpR	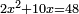$\scriptstyle2x^2+10x=48$ וזה כמו שתאמר שנים אלגוש ועשרה שרשים ‫2rישוו מ"ח אדרהמיש
compound canonical equation/squares and roots equal numbers		ax²+bx=c	חשבון_השטחים#XqGp	מרובעי' ושרשי' ישוו למספרים
compound canonical equation/squares and roots equal numbers		ax²+bx=c	תחבולות_המספר#3sJZ	ושרשים ומרובעי' שישוו למספרים
compound canonical equation/squares and roots equal numbers		½x²+5x=28	חשבון_השטחים#XyaI	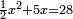$\scriptstyle\frac{1}{2}x^2+5x=28$ ואם אמרו לך חצי אלגוש וחמשה שרשים ישוו כ"ח אדרהמיש נאמר כי כאשר {{#annot: term \| #to add, #חבר \| qgPe}}חברנו{{#annotend:qgPe}} על חצי האלגוש חמשה שרשי האלגוש יהיו כ"ח אדרהמיש
simple canonical equation/squares equal numbers		ax²=c	חשבון_השטחים#kCXF	ואלאגוש ישוו מספרים
simple canonical equation/squares equal numbers		ax²=c	תחבולות_המספר#4O9C	ומרובעי' שישוו למספרים
simple canonical equation/squares equal numbers		⅓x²=27	חשבון_השטחים#sOLH	Also if you say: a third of the square is equal to twenty-seven. : $\scriptstyle\frac{1}{3}x^2=27$ וכן אם תאמר שלישית אלגו שוה ‫1vשבעה ועשרים
simple canonical equation/squares equal numbers		5x²=45	חשבון_השטחים#yMV8	Likewise, when five squares are equal to forty-five. :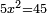 $\scriptstyle5x^2=45$ וכן חמשה אלגוש ישוו חמשה וארבעים
simple canonical equation/squares equal numbers		x²=16	חשבון_השטחים#lI7w	The squares that are equal to numbers is as a square that equals sixteen. :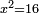 $\scriptstyle x^2=16$ והאלגוש שישוו מספרים כמו אלגו שישוה שש עשרה
simple canonical equation/squares equal numbers		⅓x²=27	תחבולות_המספר#Usmi	If one says: a third of the square is equal to twenty-seven. : $\scriptstyle\frac{1}{3}x^2=27$ ואם אמ' שלישית המרובע ישוה לשבעה ועשרים
simple canonical equation/squares equal numbers		5x²=45	תחבולות_המספר#C4DX	If one says: five squares are equal to forty-five. :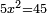 $\scriptstyle5x^2=45$ כי אם אמ' חמשה מרובעי' ישוו לחמשה וארבעי‫'
simple canonical equation/squares equal numbers		x²=16	תחבולות_המספר#fCC4	As if you are told: the square is equal to sixteen. :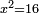 $\scriptstyle x^2=16$ כמו אם יאמרו לך המרובע ישוה לששה עשר
simple canonical equation/squares equal roots		x²=5x	תחבולות_המספר#EqMy	For example: if you are told; five roots are equal to one square. How much is the square? :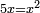 $\scriptstyle5x=x^2$ {{#annot:term\|197,1712\|lPkF}}דמיון זה{{#annotend:lPkF}} אם אמרו לך חמשה שרשי' ישוו למרובע אחד כמה הוא המרובע
simple canonical equation/squares equal roots		5x²=20x	תחבולות_המספר#WDhU	Example: if one asks: five squares are equal to twenty roots. :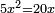 $\scriptstyle5x^2=20x$ דמיון זה אם ישאל חמשה מרובעי' ישוו לעשרים שרשים
simple canonical equation/squares equal roots		½x²=10x	תחבולות_המספר#fayj	Also, if he says: half a square is equal to ten roots. :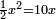 $\scriptstyle\frac{1}{2}x^2=10x$ וכן אם יאמ' חצי מרובע ישוה לעשרה שרשים
simple canonical equation/squares equal roots		½x²=10x	חשבון_השטחים#N1ie	Likewise, if it is said: half a square equals ten roots. :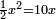 $\scriptstyle\frac{1}{2}x^2=10x$ וכמו ^כן אם יאמר חצי אלגו ישוה עשרה שרשים
simple canonical equation/squares equal roots		ax²=bx	חשבון_השטחים#bTgP	אלגוש ישוו שרשים
simple canonical equation/squares equal roots		x²=5x	חשבון_השטחים#cbxX	The squares that are equal to roots is as if you say: a square equals five roots. :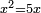 $\scriptstyle x^2=5x$ והאלגוש שישוו שרשים הוא כאלו תאמר אלגו ישוה חמשה שרשים
simple canonical equation/squares equal roots		5x²=20x	חשבון_השטחים#sJUh	As if you say: five squares equal twenty roots. :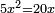 $\scriptstyle5x^2=20x$ וכמו שתאמר חמשה אלגוש ישוו עשרים שרשים
simple canonical equation/squares equal roots		ax²=bx	תחבולות_המספר#Udbo	שרשים שיהיו שוי' למרובעים

@@ Line 1: / Line 1: @@
-hello something
+{{#annotask:
+   [[category: equation/quadratic equation]]
+   [[page: author="Abū Kāmil"]]
+}}

Difference between revisions of "Search Page"

Latest revision as of 12:36, 6 September 2019

Navigation menu

Personal tools

Namespaces

Variants

Views

More

Search

Navigation

Tools