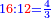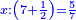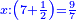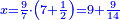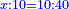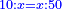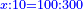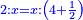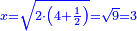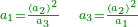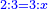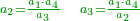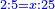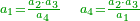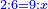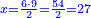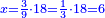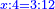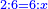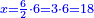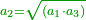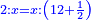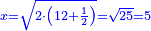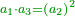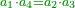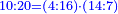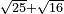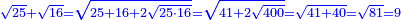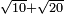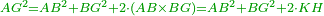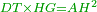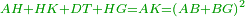Difference between revisions of "Kaufmann A506/2 – Discussion on Numbers"
From mispar
(→Chapter on the Finding a Number from a Number by Knowing Their Ratio) |
(→Chapter on the Finding a Number from a Number by Knowing Their Ratio) |
||
| Line 980: | Line 980: | ||
|colspan=3|twenty|| ||colspan=2|ten | |colspan=3|twenty|| ||colspan=2|ten | ||
|- | |- | ||
| − | |7|| || | + | |7||16|| ||14|| ||4 |
|} | |} | ||
|} | |} | ||
Revision as of 18:03, 2 June 2024
Contents
- 1 Discussion about Numbers Divided into Chapters
- 1.1 The First Method in the Introduction to the Discussion
- 1.2 The Second Introduction
- 1.3 The Third Introduction
- 1.4 Chapter on the Multiplication of Numbers by Numbers, Some of which are Knowns and Some are Unknown
- 1.5 Chapter on the Finding a Number from a Number by Knowing Their Ratio
- 1.6 The Chapter on Knowing the Root from Another Root or Roots
- 2 Appendix: Bibliography
Discussion about Numbers Divided into Chapters |
[1]המאמר במספרים נחלק לפרקי' | ||||||||||||||||||||||||||
The First Method in the Introduction to the Discussion |
הדרך הא' בהצעת המאמר | ||||||||||||||||||||||||||
| The unknown number, the knowledge of which is required, is known either through another number or numbers, or through its relation or sequence. | המספר הנעלם דרוש הידיעה יודע אם מפני מספר או מספרי' אחרים או מפני יחסו או סדורו | ||||||||||||||||||||||||||
| Through another known number: either it is equal to it, which is simple; or it is greater than it, and knowing the term that is greater than it is called addition; or it is smaller and knowing how much smaller it is, is called subtraction. | אולם מפני מספר אחר ידוע הנה או שזה שוה לו והוא פשוט או שזה יותר גדול ממנו וידיעת השעור שהוא יותר גדול ממנו ויקרא זה חבור או שהוא יותר קטן ויודע בכמה יותר קטן ויקרא מגרעת | ||||||||||||||||||||||||||
| Through other numbers: they are multiplied by each other and this is called multiplication. | אולם מפני מספרי' אחרי' שיוכפלו יחד ויקרא זה הכפלה | ||||||||||||||||||||||||||
| Through its relation, it is called ratio; or its sequence, it is called the sequential number in ratio. | אולם מפני יחסו ויקרא ערך או סדרו ויקרא המספר המסודר ביחס | ||||||||||||||||||||||||||
The Second Introduction |
הצעה שנית | ||||||||||||||||||||||||||
| The numbers that the arithmetician investigates are integers summed together called contiguous with regard to their subject. | המספרים שיעיין בם בעל חכמת החשבון הם מחוברי' מאחדי' שלמים ויקרא לאחר החלוקה בבחינת נושאו שהוא המתדבק | ||||||||||||||||||||||||||
| The mathematician investigates this also, when he thinks about the subject of the one divided into parts, whether into halves or thirds or quarters and so on. | ויעיין בו הלמודי ג"כ כשיצייר נושא האחד נחלק לחלקי' אם לחצי או לשלישי או לרביע וזולתם | ||||||||||||||||||||||||||
| The fractions are called by a name that is derived from the integers: the third is derived from three, because the greater is 3 times the smaller and the smaller is one of its three parts; the quarter is derived from the four; and so on. | ויקרא שם לשברי' נגזר מהשלמי' יקרא השליש נגזר משלש למה שהגדול ג' דמיוני הקטן הקטן חלק משלשה בו והרביע נגזר מהארבעה וכן זולתם | ||||||||||||||||||||||||||
The Third Introduction |
הצעה ג' | ||||||||||||||||||||||||||
| The arithmetic numbers are arranged in sequence according to the ratio of one to ten, since the ratio of all the units of the first rank to the units of the second rank is the same as the ratio of the units of the second to the units of the third and the same as the ratio of the units of the tenth [rank] to the units of the eleventh [rank]. | המספרי' החשבוניי' מסודרי' בהדרגה ועל יחס האחד לעשר על שיחס כל אחדי המעלה הא' לאחדי המעלה הב' כיחס אחדי השנית אל אחדי השלישית וכיחס אחדי העשירית לאחדי האחד עשר | ||||||||||||||||||||||||||
|
דמיונו שכמו ששנים במעלה השנית הם עשרה פעמי' שנים במעלה הא' כך שניים במעלה החמישית הם עשרה דמיוני לשנים במעלה רביעית | ||||||||||||||||||||||||||
| Similarly, the ranks of the sexagesimal fractions, except that the [fractions] are in a subtractive ratio and [the units] are in an additive ratio; the [fractions] are in the ratio of sixtieth to each other and [the units] are in the ratio of one to sixty. | וכן מדרגות שברי חכמי התכונה אלא שאותם הם יחס הגרעון ואלו יחס התוספת ואותם יחס אחר ששים לאחר ואלו יחס אחד מששים | ||||||||||||||||||||||||||
|
ועם זה יחס ג' דקי' לג' שניים כיחס ג' שביעיים לג' שמיניים | ||||||||||||||||||||||||||
| ולזה יפול בכל אחד מהמספרי' בחינה בבחינות המספר ומה שבו מהאחדים ובחינה במדרגתו | |||||||||||||||||||||||||||
Chapter on the Multiplication of Numbers by Numbers, Some of which are Knowns and Some are Unknown |
הפרק במכפלת מספרי' במספרי' קצתם ידועי' וקצתם נעלמי' | ||||||||||||||||||||||||||
| The unknown is called by the name of a more general species, which is a "thing". | ויקרא הנעלם בשם הסוג היותר כולל והוא דבר | ||||||||||||||||||||||||||
| If you are asked to multiply numbers whose number and value are known, by things whose number is known, but not their value: | אם ינתן לך לכפול מספרי' ידועי המניין והשעור בדברי' ידועי המניין לא השעור | ||||||||||||||||||||||||||
|
כאלו נאמר כפלנו עשרה אחדי' בד' | ||||||||||||||||||||||||||
| Here is how you answer an answer that is known from one aspect and unknown [from another]: | הנה איך אתה השיב תשובה ידועה מצד ונעלמת | ||||||||||||||||||||||||||
|
ונכפול עשרה בארבעה יעלו מ' | ||||||||||||||||||||||||||
|
ואחר בדבר יהיה דבר | ||||||||||||||||||||||||||
|
ולכן תאמר שהעולה מ' דברים כלומר הוא מ' פעמים השעור ש[כינת] אותו אתה בשם דבר מספר היה או מקובץ אחדים | ||||||||||||||||||||||||||
|
ואלו היה המכוון בדבר עשריים היה מובן השאלה תכפול עשרה אחדי' בארבעה דברים על שכל דבר הוא עשרה מספרי' | ||||||||||||||||||||||||||
|
ואם היה המכוון בדבר מאה היו מ' מאות | ||||||||||||||||||||||||||
|
וכן בכל מה שרצהו מהכוונות | ||||||||||||||||||||||||||
| Two products are needed then. | ואז צריך ב' הכפלות | ||||||||||||||||||||||||||
| Six types of combinations are created from this: | ויתחדשו בזה ו' מינים מההרכבות | ||||||||||||||||||||||||||
|
הא' שתכפול המספרי' בדברי' | ||||||||||||||||||||||||||
|
כאמרנו י' מספרי' בד' דברי' | ||||||||||||||||||||||||||
|
הב' שתכפול מספרי' במספרי' ודברי' | ||||||||||||||||||||||||||
|
כאמרנו כפול ד' מספרי' בו' מספרי' וה' דברים | ||||||||||||||||||||||||||
|
ואז צריך ב' הכפלות | ||||||||||||||||||||||||||
|
והדרך שתכפול ד' מספרי' בו' מספרי' יעלו כ"ד | ||||||||||||||||||||||||||
|
כפול ד' בה' דברים יעלו כ' דברים | ||||||||||||||||||||||||||
|
הנה המקובץ כ"ד מספרים וכ' דברים | ||||||||||||||||||||||||||
| |||||||||||||||||||||||||||
|
הג' שתכפול מספרים ודברי' במספרי' ודברי' | ||||||||||||||||||||||||||
|
ואז צריך ד' הכפלות | ||||||||||||||||||||||||||
|
כפול ד' מספרי' וג' דברים בה' מספרי' וו' דברים | ||||||||||||||||||||||||||
| |||||||||||||||||||||||||||
|
הנה נכפול הד' מספרים בה' מספרי' עלו כ' | ||||||||||||||||||||||||||
|
וד' מספרי' בו' דברי' עלה כ"ד דברים | ||||||||||||||||||||||||||
|
עוד נשוב [2]נשוב נכפול ג' דברי' בה' מספרים עלו ט"ו דברים | ||||||||||||||||||||||||||
|
נשוב נכפול ג' דברים בו' | ||||||||||||||||||||||||||
|
נקבצם עלה כ' מספרים ל"ט דברים וי"ח מרובעי הדברים | ||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||
|
הד' דרך הגרעון | ||||||||||||||||||||||||||
|
אם יאמר לך ששה מספרים בעשרה מספרים פחות ו' דברים | ||||||||||||||||||||||||||
| |||||||||||||||||||||||||||
|
הנה תכפול ששה בעשרה עלו ס' מספרים | ||||||||||||||||||||||||||
|
עוד שוב כפול ו' מספרים בששה דברים חסרים יהיו ל"ו דברים חסרים | ||||||||||||||||||||||||||
|
הנה המקובץ ששים פחות ל"ו דברים | ||||||||||||||||||||||||||
|
הה' גרעון ותוספת | ||||||||||||||||||||||||||
|
אלו אמ' לך כפול ו' מספרים וג' דברים בז' מספרים פחות ה' דברים | ||||||||||||||||||||||||||
| |||||||||||||||||||||||||||
|
הנה תכפול ו' מספרי' בז' מספרים עלו מ"ב | ||||||||||||||||||||||||||
|
כפול ו' מספרים בה' דברים חסרים עלו ל' דברים חסרים | ||||||||||||||||||||||||||
|
כפול ג' דברים נוספים בז' מספרים עלו כ"א דברים נוספים | ||||||||||||||||||||||||||
|
ג' דברים נוספים בה' דברים חסרים עלו ט"ו דברים מדברים חסרים כלומ' ט"ו מרובעי הדברים חס(ר)ים | ||||||||||||||||||||||||||
|
ונסיר מן השלשים דברים הנגרעים כ"א דברים נוספים נשארו ט' | ||||||||||||||||||||||||||
|
ויהיה כלל המקובץ מההכפלה מ"ב מספרי' ט' דברים חסרים וט"ו דברים מדברים | ||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||
| First, you should know that the result of multiplying any number by any number is a number. | וראוי שתקדם ותדע שהכפלת כל מספר בכל מספר העולה מספר | ||||||||||||||||||||||||||
| The product of a number by a thing or a number of things is a thing, or things, according to the result of multiplying the number of the things by the number. | ומספר בדבר או מניין מדברים יעלה דבר או דברים כמו העולה מכפל מניין הדברים על המספר | ||||||||||||||||||||||||||
| The product of a thing by a thing or things is a thing of a thing, that is, a square of that unknown number, or of things, according to the result of multiplying the numbers of the things by each other. | ודבר בדבר או דברים יעלה דבר מדבר כלומ' מרובע המספר ההוא הנעלם או דברים כעולה מכפל מניין הדברים קצתם בקצת | ||||||||||||||||||||||||||
| A number by an additive thing; the result is an additive thing. | ומספר בדבר נוסף יעלה דבר נוסף | ||||||||||||||||||||||||||
| By a subtractive thing; the result is a subtractive thing. | ובדבר גורע יעלה דבר גורע | ||||||||||||||||||||||||||
| A subtractive thing by an additive or a subtractive thing; the result is a subtractive thing of a thing. | ודבר גורע בדבר מוסיף או גורע יעלה דבר מדבר גורע | ||||||||||||||||||||||||||
|
הו' דרך הגרעון בכופל והנכפל | ||||||||||||||||||||||||||
|
שיאמ' כפול עשרה פחות ג' דברים בח' פחות ה' דברים | ||||||||||||||||||||||||||
| |||||||||||||||||||||||||||
| |||||||||||||||||||||||||||
|
הנה זה בד' הכפלות א' להוסיף וג' לגרוע | ||||||||||||||||||||||||||
|
נכפול י' בח' ויעלו פ' | ||||||||||||||||||||||||||
|
י' בה' דברים גורעים יהיו נ' דברים גורעים | ||||||||||||||||||||||||||
|
ח' בג' דברים גורעים יהיו כ"ד דברים גורעים | ||||||||||||||||||||||||||
|
ג' דברים גורעים בה' דברים גורעים הרי ט"ו דברי' מדברי' גורעים | ||||||||||||||||||||||||||
|
היה כלל המקובץ פ' מספרים פחות ע"ד דברים וט"ו דברים מדברים | ||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||
|
הז' גרעון ותוספת | ||||||||||||||||||||||||||
|
שיאמ' כפול עשרה מספרים ודבר על דבר פחות עשרה מספרים | ||||||||||||||||||||||||||
| |||||||||||||||||||||||||||
| |||||||||||||||||||||||||||
|
הנה זה בד' הכפלות גם כן | ||||||||||||||||||||||||||
|
והוא שתכפול הדבר הנוסף על הדבר הנוסף יעלה יעלה דבר מדבר נוסף | ||||||||||||||||||||||||||
|
הנה העשרה מספרים בי' מספרים הגורעים יהיו ק' מספרים גורעים | ||||||||||||||||||||||||||
|
תכפול י' בדבר עלו י' דברים נוספים | ||||||||||||||||||||||||||
|
שוב כפול דבר נוסף בי' מספרי' גורעי' עלו י' דברים גורעים | ||||||||||||||||||||||||||
|
כפול דבר בדבר נוסף עלה דבר מדבר נוסף | ||||||||||||||||||||||||||
|
נתן הי' דברים נוספים כנגד הגורעים ועלה כלל החשבון ק' מספרים חסרים ודבר מדבר נוסף הנה העולה דבר מדבר פחות ק' מספרים | ||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||
| As we have illustrated for integers, so this is possible for fractions. | וכמו שהמשלנו בשלמי' כן יתכן | ||||||||||||||||||||||||||
|
דמיונו כפלנו י' מספרים ושני שלישי דבר על ג' מספרים פחות ו' דברים | ||||||||||||||||||||||||||
| |||||||||||||||||||||||||||
| |||||||||||||||||||||||||||
|
הנה נכפול עשרה מספרים על ג' מספרים עלה ל' מספרים | ||||||||||||||||||||||||||
|
נכה עשרה דברים בששה דברים הגורעים יהיו ס' דברים גורעים | ||||||||||||||||||||||||||
|
נכה ב' שלישי דבר על ג' מספרים יהיו ב' דברים נוספים | ||||||||||||||||||||||||||
|
נכה ב' שלישי דבר בו' דברים גורעים יהיו ד' דברים מדברים גורעים | ||||||||||||||||||||||||||
|
נקבץ הכל אלא שנגרע הב' דברים הנוספים מהששים הגורעים נשארו נ"ח גורעים | ||||||||||||||||||||||||||
|
ולזה יהיה המקובץ ל' מספרים פחות נ"ח דברים וד' דברי' מדברים גורעים | ||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||
Chapter on the Finding a Number from a Number by Knowing Their Ratio |
[3]הפרק בהוצאת מספר ממספר מתוך ידיעת יחסו | ||||||||||||||||||||||||||
| When a number is unknown, but its ratio to another number is known and you want to find it by knowing the other number and its ratio to it: | כשהיה מספר מה נעלם ידוע היחס למספר אחר ותבקש ידיעתו מתוך ידיעת המספר האחר ויחסו לו | ||||||||||||||||||||||||||
| First, you should know that the ratio is found in three categories: | הנה ראשונה ראוי שתדע שהיחס ימצא על ג' פנים | ||||||||||||||||||||||||||
|
אם יחס מספרי והוא הבדל במספרים שלימים ידועי' | ||||||||||||||||||||||||||
|
כאלו תאמר יחס ג' לי' כיחס ג' דמיונים ואחד | ||||||||||||||||||||||||||
|
ויהיו המספרים מתיחסים כשהיה התוספת בהם שוה | ||||||||||||||||||||||||||
|
דמיונו שיחס עשרה לי"ג כיחס ק' לק"ג | ||||||||||||||||||||||||||
|
ואם יחס למודי והוא יחס בין ההבדל וכלל המספרים המתיחסים | ||||||||||||||||||||||||||
|
כאמרנו יחס ג' לעשרה כיחס ג' דמיונים ושליש | ||||||||||||||||||||||||||
| ויהיה היחס בין המספרים אחר כשיהיה ההבדל לו יחס אחד למתיחסים | |||||||||||||||||||||||||||
|
דמיונו יחס עשרה לי"ג כיחס מ' לנ"ב | ||||||||||||||||||||||||||
|
כי ההבדל בין הראשוני' הוא ג' ויחסו לעשרה כיחס כמוהו וחומשו וחצי וכן יחס מ' לנ"ב | ||||||||||||||||||||||||||
|
והג' הוא יחס נגוני | ||||||||||||||||||||||||||
| But the geometric proportion is either between two terms alone: | אולם היחס למודי יהיה לקוח אם בין שני שעורים לבד | ||||||||||||||||||||||||||
|
כאלו תאמר יחס ט' לי"ב הוא ג' רביעיות | ||||||||||||||||||||||||||
|
ויחס י"ב לי"ו הוא ד' שלישיות | ||||||||||||||||||||||||||
|
והדרך בזה כשיהיה אחד השעורים ידוע ויחס האחר אליו ידוע שיכפל היחס בשעור האחד ויצא האחר | ||||||||||||||||||||||||||
|
דמיונו שאחד השעורים ז' וחצי והאחר יחסו לו שהוא ה' שביעיות | ||||||||||||||||||||||||||
|
הנה נכפול ה' שביעיות בז' וחצי יהיה ה' וה' חלקים מן י"ד | ||||||||||||||||||||||||||
|
ואם היה ט' שביעיות | ||||||||||||||||||||||||||
|
יהיה תשעה וט' חלקים מי"ד | ||||||||||||||||||||||||||
| Or, it is between only three terms, as if you say: the ratio of one of the terms, which is the unknown, to a known term is the same as the ratio of the known to another known. | ואם יהיה בין ג' שעורים לבד כאלו תאמר יחס אחד השעורי' והוא הנעלם אל שעור ידוע כיחס הידוע אל ידוע אחר | ||||||||||||||||||||||||||
|
כאלו תאמר יחס שעור נעלם אל עשרה כיחס עשרה לארבעים | ||||||||||||||||||||||||||
|
והנה זה הנעלם אפשר שיהיה אם הראשון כמו בהמשלנו ואפשר שיהיה השני | ||||||||||||||||||||||||||
|
כאמרנו יחס עשרה לנעלם כיחס הנעלם לחמשים | ||||||||||||||||||||||||||
| Or, it is between four terms: | ואם שיהיה בין ד' שעורים | ||||||||||||||||||||||||||
|
כאלו תאמר יחס הנעלם לעשרה כיחס ק' לג' מאות | ||||||||||||||||||||||||||
|
וזה גם כן אם שיהיה הנעלם מהמספרים אשר מהקצוות או מהמספרים האמצעיים | ||||||||||||||||||||||||||
|
והראשון מאלו כבר המשלנו בו | ||||||||||||||||||||||||||
|
והשני כאמרנו יחס עשרה לנעלם כיחס ק' לג' מאות | ||||||||||||||||||||||||||
| The method is one of two options: | והדרך בזה הוא אחד משני דברים | ||||||||||||||||||||||||||
|
אם לכפול הקצוות כשהיה הנעלם האמצעי ושורש העולה הוא הנעלם וזה בג' מספרים | ||||||||||||||||||||||||||
|
כאמרך יחס שנים לנעלם כיחס הנעלם לד' וחצי | ||||||||||||||||||||||||||
|
הנה נכפול הראשון והוא ב' בג' השלישי והוא ד' וחצי והעולה והוא ט' | ||||||||||||||||||||||||||
|
נקח שורשו והוא ג' וכן השני | ||||||||||||||||||||||||||
|
וכשהיה הנעלם הוא אחד הקצוות | ||||||||||||||||||||||||||
|
נכפול האמצעי בעצמו ונחלק העולה על אחד הקצוות ויצא האחר | ||||||||||||||||||||||||||
|
דמיונו שיחס ב' לג' כיחס ג' לנעלם | ||||||||||||||||||||||||||
| |||||||||||||||||||||||||||
|
נכפול ג' על עצמו ונחלק העולה על ב' ויצא ד' וחצי וכן הנעלם | ||||||||||||||||||||||||||
| For four numbers: | ואולם בד' מספרים | ||||||||||||||||||||||||||
|
הנה אם היה הנעלם אחד האמצעיים | ||||||||||||||||||||||||||
|
הנה נכפול הקצוות ונחלק העולה על האמצעי הידוע יצא האמצעי האחר | ||||||||||||||||||||||||||
|
דמיונו יחס | ||||||||||||||||||||||||||
| |||||||||||||||||||||||||||
|
הנה נכפול ב' בכ"ה עלו נ' | ||||||||||||||||||||||||||
|
נחלקם על ה' יצא עשרה והוא הנעלם השלישי | ||||||||||||||||||||||||||
|
ואם היה אחד הקצוות הוא הנעלם | ||||||||||||||||||||||||||
|
הנה נכפול האמצעיים בעצמם ונחלק העולה על אחד הקצוות והוא הידוע יצא האחר | ||||||||||||||||||||||||||
|
דמיונו יחס ב' לו' כיחס ט' לנעלם | ||||||||||||||||||||||||||
| |||||||||||||||||||||||||||
|
הנה נכפול ו' בט' עלה נ"ד | ||||||||||||||||||||||||||
|
נחלקם על ב' עלה כ"ז וכן הרביעי | ||||||||||||||||||||||||||
| והדרך הב' מהמצאתינו שבד' שעורים שנחלק השוים הן הקרובים הידועים | |||||||||||||||||||||||||||
| ונכפול העולה על האחר על הקרוב לנעלם | |||||||||||||||||||||||||||
|
דמיונו יחס ג' לט' כיחס הנעלם לי"ח | ||||||||||||||||||||||||||
| |||||||||||||||||||||||||||
|
הנה נחלק ט' על ג' עלה שליש | ||||||||||||||||||||||||||
|
כפלנו שליש בי"ח יעלו ו' וכן האמצעי הנעלם | ||||||||||||||||||||||||||
|
בהיות הנעלם אחד הקצוות | ||||||||||||||||||||||||||
|
כאמרנו יחס הנעלם לד' כיחס ג' לי"ב | ||||||||||||||||||||||||||
| |||||||||||||||||||||||||||
|
הנה חלקנו י"ב על ג' עלה רביע | ||||||||||||||||||||||||||
|
כפלנוהו על ד' עלה אחד והוא הראשון | ||||||||||||||||||||||||||
|
ואולם בג' שעורים בהיות הנעלם אחד הקצוות | ||||||||||||||||||||||||||
|
כאמרנו יחס ב' לו' כיחס ו' לנעלם | ||||||||||||||||||||||||||
|
הנה חלקנו ו' על ב' עלה ג' | ||||||||||||||||||||||||||
|
כפלנו ג' בו' עלה י"ח וכן השלישי הנעלם | ||||||||||||||||||||||||||
|
ואם היה האמצעי נעלם | ||||||||||||||||||||||||||
|
הנה חלקנו הקצוות האחד על האחר ונקח שורש העולה והוא השני | ||||||||||||||||||||||||||
|
דמיונו יחס ב' לנעלם כיחס הנעלם לי"ב וחצי | ||||||||||||||||||||||||||
| |||||||||||||||||||||||||||
|
כפלנו ב' בי"ב וחצי עלה כ"ה | ||||||||||||||||||||||||||
|
לקחנו שורשו והוא ה' וכן הוא האמצעי | ||||||||||||||||||||||||||
| The reason for all these methods: | והסבה בכל אלו הדרכים | ||||||||||||||||||||||||||
|
אם לראשון הנה לפי שהיו השעורים המתיחסים שיור העולה מהכאת הראשון בשלישי כהכאת | ||||||||||||||||||||||||||
|
ובהיותו בין ד' מספרים היה הכאת הראשון באחרון כהכאת השני בשלישי | ||||||||||||||||||||||||||
| Hence, when the unknown is the mean, the product of the mean by itself is known since it is the same as the product of the first by the last. | |||||||||||||||||||||||||||
| Because, when the ratio is between four terms, the product of the second by the third is known and the unknown is one of the means. | כי הכאת השני בשלישי ידוע כשהיה היחס בין ד' שעורים [4]שעורים והנעלם אחד מהאמצעים | ||||||||||||||||||||||||||
| Or, the product of the first by the last is known, when the unknown is one of the extremes. | או שטח הראשון באחרון ידוע כשהיה הנעלם אחד הקצוות | ||||||||||||||||||||||||||
| When a product is known and one of its factors is known, the other factor is also known by division, as above: | וכשהיה שטח מה ידוע ואחד צלעיו ידועים הנה הצלע האחר גם כן ידוע בדרך חלוקה כמו שקדם | ||||||||||||||||||||||||||
| By dividing the product of the mean by itself, or of the means, by one of the extremes, the other results. | הוא כשנחלק שטח האמצעי בעצמו או האמצעים על אחד הקצוות יצא האחר | ||||||||||||||||||||||||||
| For four terms: if we divide the product of the extremes by one of the means, the other results. | ואם נחלק שטח הקצוות על אחד האמצעיים יצא האחר בד' שעורים | ||||||||||||||||||||||||||
| For three [terms]: when we find its root, it is the factor of the square that is equal to the product formed by the multiplication of the extremes. | ובג' כשנמצא | ||||||||||||||||||||||||||
| Regarding the second method, since the ratio of the unknown to the known is known, because it is the same as the ratio of the known to another known, then the ratio between the two knowns is itself the same as the ratio of the unknown to the known. Therefore, when the ratio is multiplied by the known the result is the unknown. | ואולם לדרך הב' כי אחר שהיה | ||||||||||||||||||||||||||
| The ratio between six terms: | והיחס בין ששה שעורים | ||||||||||||||||||||||||||
|
שתאמר יחס עשרה לעשרים מורכב מיחס ד' לי"ו ומיחס י"ד לז' | ||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||
The Chapter on Knowing the Root from Another Root or Roots |
[5]הפרק בידיעת השורש מפני שורש אחר או שרשים | ||||||||||||||||||||||||||
| It is divided into five investigations: | ויחלק לה' דרושים | ||||||||||||||||||||||||||
|
הא' מפני קבוץ השרשים | ||||||||||||||||||||||||||
|
הב' מפני כפלם | ||||||||||||||||||||||||||
|
הג' מפני חלוקתם | ||||||||||||||||||||||||||
|
הד' מפני סדרם | ||||||||||||||||||||||||||
|
הה' מפני יחסם | ||||||||||||||||||||||||||
The First Investigation |
הדרוש הא' | ||||||||||||||||||||||||||
| We are looking for [the sum of two roots]. | בקשנו השורש העולה מחבור ב' שרשי' | ||||||||||||||||||||||||||
|
כאלו תאמר חברנו שורש כ"ה בשורש י"ו ונבקש השורש המקובץ | ||||||||||||||||||||||||||
|
הנה הדרך בזה שתחבר השני מרובעים יחד ועלה מקובצם מ"א | ||||||||||||||||||||||||||
|
הכה שני המרובעים קצתם בקצת עלה ת' | ||||||||||||||||||||||||||
|
קח שני שרשיו והם מ' | ||||||||||||||||||||||||||
|
תקבצם עם מחובר השני מרובעי' שהוא מ"א עלה פ"א | ||||||||||||||||||||||||||
|
קח שרשו והוא ט' והוא כמו מקובץ שורש י"ו בשורש כ"ה | ||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||
|
וכזה כשבקשנו חבור שורש | ||||||||||||||||||||||||||
|
חברנו עשרה בעשרי' עלו ל' | ||||||||||||||||||||||||||
|
כפלנו י' בכ' עלה מאתים | ||||||||||||||||||||||||||
|
|||||||||||||||||||||||||||
|
כפלנום עלה כ"ח וב' נעלמים | ||||||||||||||||||||||||||
|
קבצנום עם ל' עלה נ"ח וב' נעלמים | ||||||||||||||||||||||||||
|
ושורשו הוא הדרוש | ||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||
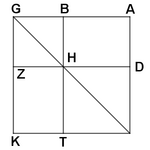
| The reason is that we suppose the two roots are lines AB and GD; the square of AB is DT and the square HG is the square of BG.
|
והסבה בזה שנניח השני שרשי' קוי א"ב ג"ד ומרובע א"ב ד"ט ומרובע | ||||||||||||||||||||||||||
|
|||||||||||||||||||||||||||
| Since the square AG is equal to [the sum of] the squares AB and BG plus double the product of AB by BG, which is double the area of KH. | הנה לפי שמרובע א"ג שוה לשני מרובעי א"ב ב"ג וכפל שטח א"ב בב"ג שהוא כמו כפל שטח כ"ה | ||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||
| Also, the area of AH is mean in the ratio between the two squares HT and HG.
|
ושטח א"ה אמצעי ביחס בין שני מרובעי ד"ט וה"ג | ||||||||||||||||||||||||||
| Therefore, the product of DT by HG is the same as the product of AH by itself.
|
היה כפל מרובע ד"ט בה"ג כמו כפל א"ה בעצמו | ||||||||||||||||||||||||||
| So, when we multiply the square DT by HG, then extract the root of the result, that root is equal to the area of AH.
|
הנה כשכפלנו מרובע ד"ט בה"ג ולקחנו שורש העולה היה השורש ההוא שוה לשטח א"ה | ||||||||||||||||||||||||||
| When we multiply it [by it self, the result is] the two surfaces AH and HK. | וכאשר הכפלנוהו על שני שטחי א"ה ה"כ | ||||||||||||||||||||||||||
| When we sum them with the two known squares DT and HG, the total is the square AK, whose root is known, which is the sum of AB and BG.
|
וכאשר קבצנום עם שני מרובעי ד"ט ה"ג הידועים היה כלל מרובע א"כ ידוע שרשו המחובר מן א"ב ב"ג ידוע | ||||||||||||||||||||||||||
The Second Investigation |
הדרוש הב' | ||||||||||||||||||||||||||
| We are looking for the result of subtraction of two roots from each other. | בקשנו העולה מגרעון שני שרשים האחד מהאחר | ||||||||||||||||||||||||||
|
כאלו תאמר בקשנו לגרוע שורש | ||||||||||||||||||||||||||
Appendix: Bibliography
Manuscript:
- Budapest, Magyar Tudományos Akadámia, Ms. Kaufmann A 506/2 (IMHM: f 14991), ff. 114-118 (15th century)

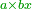
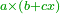
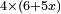
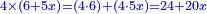
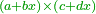
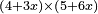
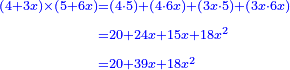

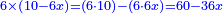
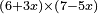
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle\left(6+3x\right)\times\left(7-5x\right)&\scriptstyle=\left(6\sdot7\right)+\left[6\sdot\left(-5x\right)\right]+\left(3x\sdot7\right)+\left[3x\sdot\left(-5x\right)\right]\\&\scriptstyle=42-30x+21x-15x^2\\&\scriptstyle=42-\left(9x+15x^2\right)\end{align}}}](/mediawiki/images/math/4/e/0/4e0edb80553ecff04e7bdebf56203251.png)
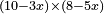
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle\left(10-3x\right)\times\left(8-5x\right)&\scriptstyle=\left(10\sdot8\right)+\left[10\sdot\left(-5x\right)\right]+\left[8\sdot\left(-3x\right)\right]+\left[\left(-3x\right)\sdot\left(-5x\right)\right]\\&\scriptstyle=80-50x-24x-15x^2\\&\scriptstyle=80-74x+15x^2\end{align}}}](/mediawiki/images/math/7/b/1/7b1e6732b11581e8dfe05fca0b65237e.png)

![\scriptstyle{\color{blue}{\begin{align}\scriptstyle\left(10+x\right)\times\left(x-10\right)&\scriptstyle=\left[10\sdot\left(-100\right)\right]+\left(10\sdot x\right)+\left[x\sdot\left(-10\right)\right]+\left(x\sdot x\right)\\&\scriptstyle=-100+10x-10x+x^2\\&\scriptstyle=-100+x^2=x^2-100\end{align}}}](/mediawiki/images/math/c/7/2/c724dd0794664d825f22627e3e412352.png)
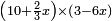
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle\left(10+\frac{2}{3}x\right)\times\left(3-6x\right)&\scriptstyle=\left(10\sdot3\right)+\left[10\sdot\left(-6x\right)\right]+\left(\frac{2}{3}x\sdot3\right)+\left[\frac{2}{3}x\sdot\left(-6x\right)\right]\\&\scriptstyle=30-60x+2x-4x^2\\&\scriptstyle=30-58x-4x^2\end{align}}}](/mediawiki/images/math/b/d/7/bd795942906b597817bd11893d6d27b6.png)
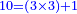
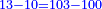

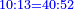
![\scriptstyle{\color{blue}{10:13=1:\left[1+\frac{1}{5}+\left(\frac{1}{2}\sdot\frac{1}{5}\right)\right]=40:52}}](/mediawiki/images/math/4/e/7/4e785b1932d5a4b4d5be4ecec2cb0e11.png)