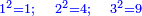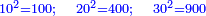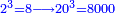|
|
| Line 1,415: |
Line 1,415: |
| | |- | | |- |
| | |Henceforth some specific qualities of the nature of number will be presented by way of a tale and description | | |Henceforth some specific qualities of the nature of number will be presented by way of a tale and description |
| − | |style="text-align:right;"|ואחר שהגענו לזה המקום נביא קצת '''סגולות פרטיות מטבע המספר''' בדרך הגדה וספור | + | |style="width:45%; text-align:right;"|ואחר שהגענו לזה המקום נביא קצת '''סגולות פרטיות מטבע המספר''' בדרך הגדה וספור |
| | |- | | |- |
| | |Not as the way used by <span style=color:blue>'''Euclid'''</span> in <span style=color:blue>'''the ''Elements'', books 7-9'''</span>, because the number does not require this, since the practical counting verifies any hypothetical proposition, even there the reader will not rest until checking it through the counting test, hence you find Euclid at the end of every proposition brings a numerical example, and not just for the numerical propositions, but also for the geometric propositions. Every matter that could be examined with numbers is translated to numbers, as in most of the propositions of the <span style=color:blue>'''second book''' of '''Euclid's ''Elements'''''</span> | | |Not as the way used by <span style=color:blue>'''Euclid'''</span> in <span style=color:blue>'''the ''Elements'', books 7-9'''</span>, because the number does not require this, since the practical counting verifies any hypothetical proposition, even there the reader will not rest until checking it through the counting test, hence you find Euclid at the end of every proposition brings a numerical example, and not just for the numerical propositions, but also for the geometric propositions. Every matter that could be examined with numbers is translated to numbers, as in most of the propositions of the <span style=color:blue>'''second book''' of '''Euclid's ''Elements'''''</span> |
Introduction
|
|
| The issue discussed in this chapter as the purpose of this wisdom [= mathematics/arithmetic]
|
דע שזה השער הוא הנכבד שבזאת החכמה וכאלו הוא התכלית בה
|
| Due to the importance this issue the Ancients wrongly assumed that the numbers are transcendent and are the beginning of the perceptible existence
|
ולרוב מעלת זאת החקירה טעו בה הקדמונים והניחו מספרים נבדלים ושמום התחלות המציאות המוחש
|
- For they have found that quantity is said with regard to all material and spiritual things
|
וזה שמפני שהם מצאו הכמה נאמר בכל הדברים גשמיים או רוחניים
|
- In relation to God, it is said: the extent of perception and power, or endless power etc.
|
וזה שבאלוה יאמר גודל ההשגה והיכולת או אין תכליות ביכולת ודומה לזה
|
- Endless is an unlimited quantity whether in measure or in number
|
ואין תכלית הוא כמה בלתי מוגבל בין בשיעור בין במספר
|
- In the separate intellects: there is a plurality and counting with regard to cause and effect, or existence and essence
|
וכן מצאו בשכלים מהנפרדים רבוי וספירה לפחות מצד עלה ועלול או מציאות ומהות
|
- Most of the perceptible existences preserve limited relations
|
וג"כ מצאו רוב הנמצאות המוחשות שומרות יחסים מוגבלים
|
- The measure of the bodies of the stars, the thickness of their spheres, and their eccentricity
|
כענין בגודל גרמי הכוכבים ועובי גלגליהם ויציאת מרכזיהם
|
- The eight limited wheels in the universe
|
וכן בעגולים המוגבלים בכדור הח'
|
- The thickness of the elements
|
וכן בעובי גרמי היסודות
|
- The organs of the animals such as the joints of their organs
|
וכן באברי הב"ח כמו פרקי איבריהם
|
- The extremes of the species and the quantity of its individuals
|
וקצוות המין בגודל אישיו כמו שנעיר על קצת מאלו בזה השער
|
| Therefore this matter caused them to praise the number until they referred to it as a beginning, and they did so also in relation to quantity
|
הביאם הענין להגדיל המספר עד שייחסו אליו היותו התחלה וכן עשו בשעור
|
| But, according to the author of the text, this assumption is a mistake
|
כמו שאמרנו אמנם שזה הסברה טעות
|
| The number is incident of the counted and therefore cannot be a beginning
|
ושהמספר מקרה בספורים מה שבמקרה אי אפשר לשומו התחלה אין כאן מקומו
|
- Relying on the words of Aristotle = "The Philosopher" in a few instances in his Physics, and many instances in the Metaphysics
|
וכבר האריך בזה הפילוסוף בקצת מקומות מהשמע ובהרבה ממה שאחר הטבע
|
- As well as his commentators and some later Greek, Arab and Christian writers
|
והרחיבו בו מפרשי ספריו וכמה מחברים אחרונים יווניים וערביים ונצרים
|
| Nevertheless, there are indeed wondrous qualities and exceptional natures in number
|
ועל האמת יש במספר סגולות נפלאות וטבעים משונים
|
| The reasons of some of them are visible, the reasons of others are hidden, though they themselves are known to exist, but most are hidden themselves as well as their reasons
|
מהם גלויי הסבות ומהם נעלמי הסבות אבל הם ידועי המציאות ורובם שנעלמו מאתנו אלו ואלו
|
| The purpose of the soul to apprehend the natural matters
|
ואין ספק שכל ענין וענין מענייני הטבע הכוללים כשתשיגהו הנפש ותדעהו תשמח ותתענג בזה מאד לפי שזה תכליתה ולזה הכינה הכח האלהי לקבול פיתוחי הנמצאות וציוריהן
|
- Reference to Aristotle's On the Soul, III, (4, 429b30-430a2) - the image of the soul as a writing-table
|
ומפני זה אמר הפילוסוף בשלישי מן הנפש שהיא כמו הלוח החלק המקבל כל ציור וכתיבה
|
- Reference to Al-Ghazālī's Maqāṣid al-Falāsifa (The Aims of the Philosophers, the logical part) - the image of the soul as a polished mirror
|
ואלגזאלי אמר בתחלת ספרו בכונות שהיא כמו מראה זכה מלוטשת מקבלת דמות המציאות כלו כל עת שלא יחול מסך בינה ובינם בכל או בקצת
|
| As the soul benefits from the knowledge of the properties of natural things, even though it does not know their reasons, so it would benefit from knowing the qualities of the discontinuous and continuous quantity even though it will not know the reasons of some of them
|
וכמו שתהוה הנפש מידיעת סגולות העצים והאבנים ואברי הב"ח המתדמים והכליים אע"פ שתסכל ברובם הסבות תתענג הרבה בהשיגה המציאות ותצטער בסכלה הרבה ותצטער יותר כשתסכל אלו ואלו כן כשתדע הנפש סגולות הכמה המתחלק והמתדבק אע"פ שתסכל בקצתם הסבות תתענג הנפש תענוג גדול במה שהשיגה
|
- Reference to the saying of an Arab sage who praise the knowledge of the natures of the existences without knowing their reasons
|
ולזה אמר אחד מחכמי ישמעאל מי יתן ונדע טבעי כל הנמצאות ונסכל סבותיהם
|
| The one who knows the characteristics and properties of number:
|
ונשוב למה שהיינו בו ונאמר שמי שידע טבעי המספר וסגולותיו
|
- 1) Knows many of the characteristics of the existance, which should always be known
|
ידע הרבה מטבעי המציאות אין ראוי שיוסכלו לשלם
|
- 2) Deduces some precious guidelines concerning the world, God, the angels, the spheres, the soul, and the lower existences - of which not much is known
|
ועל הכונה הב' יקח מהם כמה הערות יקרות בעולם באלוה ובמלאכי' ובגלגלים ובנפש ובנמצאות השפלות ואנחנו בלא ספק נדע מעט מהם ונסכל הרבה ואף גם זאת במעט אשר נודע לחכמים ואמרו בו כמה רמזים יקרים משמחי לב ומאירי נפש
|
| The same goes to geometry - there are wondrous qualities in geometry that testify to valuable secrets
|
ודע שזה הענין כן הוא בהנדסה ר"ל שיש בה סגולות נפלאות יעי[דנו] על סודות נכבדים
|
| God has set these two [= arithmetic and geometry] as an analogy and example to the whole existence
|
וכאלו ב' אלה הטבעים שמם האלוה ית' על הכונה השנית דמיון ודוגמא למציאות כולו
|
| The author states that his discussion is based on what he has found in the words of the previous scholars
|
ואחר שהקדמנו זה נתחיל לדבר בזה כפי מה שמצאנו לחכמים לפנינו
|
| He declares that he does not elaborates and does not refer to implausible remarks of the predecessors, but summarizes as much as possible without leaving out what is necessary
|
מעט שהושקף לנו מבלתי שנעמיק ונטה אל קצת הערות דחוקות זכרום קצת אנשים מצורף למה שהסכמנו לקצר כפי כחנו מבלתי שנשמיט ההכרחי
|
|
|
Section One - Discussion on the Numbers One - Ten
|
|
One
|
|
| The numerical one testifies to issues related to God –
|
ומעתה נתחיל ונאמר שמהאחד המספריי נוכל להכיר כמה עניינים בבורא
|
- It cannot be multiplied [1×1=1; a×1=a], divided [1÷1=1; a÷1=a] or changed, and therefore cannot be increased when multiplied by itself
|
מהם שכמו שהאחד המספרי מצד שהוא א' לא מתרבה ולא מקבל חלוק ושנוי ולזה לא יתרבה בהכפלו בעצמו כן הבורא ית' שהוא אחדות אחת יותר פשוט לא יתואר בצד מן הצדדים ברבוי בעצמו רק ביחס השלמיות אליו או בצירוף פעולתו בנמצאות
|
- It is in itself in actu and in number in potentia
|
וכמו שהא' המספרי הוא בעצמו בפעל ובמספר בכח כן האלוה ית' הוא בעצמו בפעל גמור אחר שהוא עלת הכל ונבדל והוא בכח בכל אחד מן הנמצאות
|
- The author mentions a saying of a christian sage according to whom God offers himself to every being
|
ומזה הצד אמרו החכמים שהבורא בכל ולזה אחד מהחכמים כששאלו תלמידו אנה הוא האלוה השיבו אנה אינו ר"ל שהכח האלהי מצוי בכל נמצא כפי מה שבטבעו שיקבל ממנו וזה הוא מה שאמר א' מחכמי הנוצרים שהאלוה מתנדב עצמו לכל מצוי
|
- It is the cause and beginning of number but not a number itself; the number exists by it and it is a part of the number, but the number is not a part of it
|
וכמו שהא' המספרי עלת המספר והתחלתו ואינו מספר ולא יצדק בו גדר המספר ובו קיום המספר ולא עד שיחולק המספר בחלוקו והוא לא יחולק בהסתלק המספר כן האלוה האחד הוא עלת כל הנמצאות כלן והתחלת היותן וקיומן ואינו משאר הנמצאות ולא חלק מהן אבל הוא נבדל מעלוליו עם היותו עלה ובהסתלקו יסתלקו שאר הנמצאות ולא יסולק הוא בהסתלקן
|
- It has only one side – it is the beginning of counting and the multitude continues from it to infinity in potentia
|
וכמו שהאחד המספריי אין לו רק פאה א' לפי שהוא גבול הספירה וממנו ימשך הרבוי עד לאין תכלית בכח וכלה הוא בכל גבול הנמצאות אין אחריו כלום לא בפעל ולא במחשבה
|
|
|
וזה שאפי' המחשבה אינה הולכת לאין תכלית אבל מכח אלהותו ירבו ויתמעטו הנמצאות ויאצלו עד תכליתן בהדרגה במין ולאין תכלית באישים באים זה אחר זה בכח
|
- The author rejects the common saying that one is a root, a square, a cube, or a triangular
|
ומה שאמרו קצת אנשים שהאחד שרש ומרובע ומעוקב ומשולש הוא טעות
|
- since the numerical one is devoid of all subjects outside the essence and thus has no multitude or division
|
וזה שהאחד המספריי לקוח בשכל מופשט מכל נושא חוץ לנפש ומצד שהוא כן אין רבוי בו בשום פנים ואיך א"כ יתואר במה שיסבול ההחלק והרבוי כמו מרובע ומעוקב שהם דברים מתחלקים בכח לאין תכלית ואם נאמר שהאחדות המונח חוץ לנפש כמו אמה וזרת שומרים אותו האחדות ברבוע ועקוב אנו אומרים שזה הבל שכבר נתרבו אלו באלכסוניהם
|
- Despite all the above, even though the characteristics of the numerical unity are similar to the nature of divinity, the possibility that God will be described by a numerical unity is denied –
|
ואם ישאל שואל למה לא יתואר הבורא ית' באחדות המספריי אחר שטבעיו דומים לטבע האלהות
|
- These similarities are not founded on one nature
|
נשיב לו שאלו הדמויים אינם כלם ולא רובם על טבע אחד
|
- Some of the properties of numbers are shared also by one, in most cases it shares the characteristics of odd numbers [Note: here a hint is given for two chapters which appeared in the original text before the present chapter but are missing in the survived version]
|
ועוד שהאחדות המספריי ישיגהו שלפעמים ילבש מלבוש נכרי וישתתף לריבוי ר"ל למספר בקצת טבעיו כמ"ש בקצת מקומות מב' שערים שעברו ויתבאר יותר בזה השער כשנדבר בסגולות הפרטיות וזה שיש טבעים שישיגו המספרים והאחד עמהם וברוב המקומות טבעו טבע הנפרד
|
| The fact that one shares the characteristics of numbers at times, but in other occasions not - is among of the things related to number whose reason is very mysterious
|
וזה א' מהדברים הנמצאים במספר שסבתו נעלמת מאד ר"ל היות האחד פעם טבעו טבע מספר ופעם לא
|
| Hence, the unity of God is different than the numerical unity
|
יתבאר א"כ מזה שאחדות האלוה מתחלף מהאחדות המספריי
|
| The unity of God does not cease in any aspect and relation, it is a simple unity permanent forever almost inaccessible for the intellect due to its depth and subtlety
|
וזה שאחדות האלוה לא יפסד בשום בחינה וצירוף אבל הוא אחדות פשוט שמור לעולם כמעט שיבצר מן השכל לעומקו ודקותו
|
Monad in the existences
|
|
| The worthy existences are united in receiving the quality of unity - their species exists as one individual
|
וכמו שהאחדות המספריי יכלול כל המעלות הנזכרות ויותר הרבה נתיחדו הנמצאות הנכבדות לקבל טבע האחדות כפי כחן והיה מינם מתקיים באיש א' כמו האלוה ית' והשכלים הנפרדים לפי שהם יתחלפו ב[מ]יניהם במין והשמש והירח יתחלפו כלם כן וזה שאחר שיש להם פעולות מתחלפות במהות המקבל א' בעצמו הנה הם בלא ספק יתחלפו במהות ובמין אם כן כל א' מהם יחידי במינו
|
| The universe as a whole is one from this aspect - as explained by Aristotle, On the Heavens, I.8, 277b8-13
|
גם העולם בכללו מצד זה א' לבד כמו שביאר הפילוסוף בראשון מהשמים והעולם
|
|
|
Two
|
השנים
|
- The beginning of the numerical multitude
|
תחלת הרבוי המספריי
|
- The beginning of the existent multitude
|
וכן תחלת הרבוי הישיי
|
- The separate intellect, which follows the unity of God, has duality – it is composed of
|
וזה שאחר אחדות האלוה הוא השכל הנפרד ואין בו רק השניות והוא העלול הא' שיש בו הרכבה ויש בו הרכבה
|
|
מעלה ועלול
|
|
וממציאות ומהות
|
- Since it is an effect, for every existence is an effect, the existence is an accident in it, so the accident and the holder of the accident are the same, whereas in God, though it is an effect, the existence and the essence are absolutely one
|
לפי שהוא עלול וזה שכל נמצא עלול המציאות מקרה בו והמקרה ובעל המקרה שוים אבל האלוה לפי שאינו עלול המציאות והמהות בו אחד לגמרי
|
Properties of the number two
|
|
- Of the nature of two is that its sum is as its product [
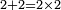 ], i.e. that the multitude of the first effect is not absolute multitude, but only in comparison to a multitude of essences, therefore it is similar to the nature of the number two, whose product does not exceed its sum, unlike all the numbers that follow it, whose products exceed their sums [for every ], i.e. that the multitude of the first effect is not absolute multitude, but only in comparison to a multitude of essences, therefore it is similar to the nature of the number two, whose product does not exceed its sum, unlike all the numbers that follow it, whose products exceed their sums [for every  : :  ] ]
|
ומטבע השנים שמחברתו כמערכתו ר"ל שרבוי העלול הראשון אינו רבוי גמור רק בהצטרף לו רבוי [מהויות][1] ולזה הוא דומה לטבע השניות המספרי שמערכתו לא יותיר על מחברתו וזה בחלוף כל המספרים שאחריו וזה שכולם יעדיף מערכתם על מחברתם
|
- As if it is mean between the nature of unity and the multitude, thus, in its nature, it is situated between one and three.
|
וכאלו הוא ממוצע בין טבע האחדות והרבוי ולזה בטבעו הוא מונח בין האחד והשלשה
|
- Since the sum of one is greater than its product [
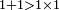 ] ]
|
וזה שהאחד מחברתו יותר ממערכתו
|
- And vice versa for three [
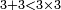 ] ]
|
והג' בהפך
|
- Therefore two has a moderate nature and similarly is the first effect that is not entirely one and not completely multiple
|
ולפי שהשנים ממוצע ביניהם יש לו טבע ממוצע בין עניין העלול הראשון שאינו א' לגמרי ולא רב לגמרי
|
|
|
והנה השנים הזוג הראשון
|
Dyad in the existences
|
|
| Some general things in the existence:
|
וכמה דברים כוללים במציאות
|
|
|
והנה השנים הזוג הראשון שירוץ מספרם בשנים בין בשכלים בין בגלגלים בין ביסודות והמורכב מהם
|
- The aspect of duality in God: one from the aspect of itself, two from the aspect of its other.
|
באלוה בחינת שניות האחד בבחינת עצמו השני בבחינת זולתו
|
- As it is a cause or actor
|
כמו שהוא עלה או פועל מזה שהתחלות הדברים המוחשים זוג השניות
|
|
|
אם בגשמים היסודים החומר והצורה
|
|
|
ואם ברקיעים הנושא והצורה
|
- types of limitations/parallels [?]
|
וכל מי המגבילות זוג השנים
|
- affirmation, negation (Aristotle, De interpretatione I.4, 16b)
|
והגבלת האמתיות ובשוללות ולזה היא היותר קודמת שבמושכלות כמו שביאר הפילוסוף בשני ממה שאחר
|
- The truth and false are two.
|
והאמת והשקר שנים
|
- The parts of the time are two: past and future; since the present is unlimited and not enduring.
|
חלקי הזמן שנים עבר ועתיד וזה שההוה בלתי מוגבל ולא קיים
|
- The life and death are two.
|
החיים והמות שנים
|
- The potentiality and the actual are two.
|
הכח והפועל שנים
|
- The change is by two: causing and accepting, i.e, receiving.
|
השנוי בשנים הפעל וההתפעלות ר"ל הקבול
|
- The [types of] bodies are two: the earthly and heavenly.
|
הגופות שנים היסודי והרקיעי
|
- The earthly [types of bodies] are two: simple and compound.
|
היסודיי שנים פשוט ומורכב
|
- The heavenly [types of bodies] are two: planet and star; because they differ in essence, since the planet is transparent, while the star is not, and the planet moves in its essence, whereas the star by accident.
|
הרקיעיי שנים הגלגל והכוכב וזה שהם מתחלפים במהות לפי שהגלגל ספירי והכוכב לא והגלגל מתנועע בעצם והכוכב במקרה
|
- The movements of the planets are two lengthwise – west and east; and two breadthwise – north and south.
|
תנועות הגלגלים הגלגלים בארך שנים ימה וקדמה וברוחב שנים צפונה ונגבה
|
- The planets differ and associate in their natures two-by-two as the astrologers said:
|
הגלגלים יתחלפו ישתתפו בטבעיהם שנים שנים כמו שיאמרו האצטגנינים
|
- Two of them are luminaries.
|
וזה שמהם שנים מאורות
|
- Two are beneficial: Jupiter and Venus.
|
ושנים מצליחים צדק ונגה
|
- Two are malefic: Saturn and Mars.
|
ושנים מזיקים שבתי ומאדים
|
- Mercury has no permanent nature, but it is changeable according to their sayings.
|
וכוכב אין לו טבע מוגבל אבל הוא לפי דבריהם מתהפך
|
- The nature of the genus is two: male and female; there is no third that is mean between them, only by natural error, as the one whose sex is indeterminate and the hermaphrodite
|
טבע הסוגיות בשנים זכר ונקבה ואין ביניהם שלישי ממוצע רק בשגיאת הטבע כמו הטומטום והאנדרוגינוס וזה אשר אמרנו בנולד מכמוהו במין אבל במגילד מהעיפוש אע"פ שהוא ממה שבטבע הנה אינו טבעי
|
- The types of the composed organs are two: homogeneous and organic.
|
מיני האברים המורכבים שנים מתדמה וכליי
|
- The types of analogies are two: equality and difference.
|
סוגי ההקשה שנים השתוף וההבדל
|
- The living species are two: actor and intelligent.
|
מיני החיים שנים פעליים ומחשביים
|
- The types of laws are two: natural and punitive (Aristotle, ?)
|
מיני הנימוסים שנים טבעי וקנסי כמו שביאר הפילוסוף בשני מס' ההליכה
|
- durations of existence: in time, in eternity
|
המשכות הנמצאות בשנים בזמן או בנצחות
|
- relations between the beings: agreement, difference (Ikhwān al-Ṣafā, Jābir ibn Hayyān)
|
בחינת הנמצאות אלו עם אלו בשנים והם ההאותות והחלוף וזה סוג כולל יכנסו תחתיו כל מיני טבעי הנמצאות כמו שביארו מחברי אחואן אלצפא וגאבר בן חיאן מלמד הכימיא בספרו בסגולות הדברים
|
- kinds of excellences: intellectual and moral (Aristotle, Nicomachean Ethics, II.1, 1103a14-25)
|
סוגי האשור שנים מחשביי ומדותיי כמו שביאר הפילוסוף בראש המאמר [א]ב' מהמדות
|
|
|
Three
|
השלושה
|
Properties of the number three
|
|
|
|
הוא ראשון למספרים הנפרדים
|
- Concludes the three kinds of numbers – one, even and odd
|
ובו נשלם כל טבע המספר וזה שבו האחד ושני מיני הריבוי שהם הזוג והנפרד
|
Triad in the existences
|
|
|
|
וכמו שהשלשה בסדור המספר אחר שנים כן בהשתלשלות המציאות טבע השלוש מגיע אחר השניות
|
- God is described as: the form, the act, and the purpose of the world
|
וזה שאחר שנניח האלוה ושהוא ממשיך המציאות מאתו יתואר בשהוא צורת העולם ופועלו ותכליתו
|
- According to Ibn Rushd, in the second book of his epitome on the Metaphysics, these three are one, and do not indicate plurality in God
|
ושלשה אלו הם א' לא יתנו רבוי בעצמו ית' כמו שביאר בן רשד בסוף השני מקצורו למה שאחר
|
- According to Aristotle in natural things these three are also one in subject and three in observation
|
וגם בדברים הטבעיים הוא כן כי הצורה והפועל והתכלית א' בנושא שלשה בבחינה כמ"ש ארסטו בשני מהשמע
|
- God is descibed also as: intellect, the endowed with intelligence, intelligence
|
וכן יתואר הבורא בשלוש שהוא שכל ומשכיל ומושכל
|
- According to the great sages these three do not indicate plurality in God
|
ולא יביאו אל הרבוי כמו שביארו גדולי החכמים
|
- Thus, Aristotle said (On the Heavens, I, 268a1-268b10) that because three has two ends and a middle it is whole and complete and therefore God should be magnified by this number, in accordance with the act of nature as a law
|
ולזה אמר ארסטו בתחלת הראשון מספר השמים כשביאר שהג' כל ושלם אחר שיש לו ב' הקצוות ואמצעי שראוי מפני זה שנגדיל האלוה בזה המספר כדי שנמשך לפעל הטבע ויהיה זה כאלו הוא תורה לנו
|
- three prayers (based on Ibn Rushd saying)
|
ובן רשד המפרש פי' בו מספר התפלות והקרבנות ואולי זאת היתה כוונת מיסדי תפלותינו שהם שלש אנחנו הישראלים
|
- attributes of God: Wisdom, Power, Will
|
תארי הבורא בשלמות שלשה חכמה ויכולת ורצון
|
- what follows the second cause: the first orb, its essence, and the mover of the second orb
|
והעלה השניה ממשיכה אחריה ג' והם הגלגל הראשון ונפשו ומניע הגלגל השני
|
- properties of the heavenly bodies: in each of the seven spheres many orbs are moving one star; in the eighth sphere one orb moves many stars; in the ninth sphere there is no star
|
ובגרמים השמימים טבע השלוש וזה ששבעת הכדורים בכל א' גלגלים רבים להניע כוכב א'
ובשמיני גלגל א' יניע אלף וכ"ב כוכבים ובט' אין בו כוכב
|
- longitudinal motions of the stars
|
ומצד אחר כוכבי הרקיע השמיני תנועתם פשוטה מתדמה סביב מרכז העולם ובמאורות בלתי מתדמה ולא סביב מרכז העולם אבל ישיגם נזורות ובה' כוכבי הנבוכה ישיגם הנזורות הנה נבדלו הגופים הרקיעים בג' טבעים בתנועתם באורך
|
- horizontal motions of the stars
|
ומצד אחר בתנועת הרחב וזה שהשמש אין לו תנועה ברוחב מאזור המזלות והירח יתנועע ברוחב בכל גלגלו הנוטה ברוחב קיים הנטיה ובחמשת הכוכבים גלגלם הנוטה בלתי קיים
|
- eccentric sphere or epicycle; eccentric sphere and epicycle; neither
|
ומצד אחר השמש לפשיטותו יספיק בו יציאת המרכז או גלגל הקפה והששה הנשארים צריכים לשניהם וכוכבי שבת אין בהם לא זה ולא זה
|
- solar eclipse; lunar eclipse; neither
|
ומצד אחר השמש תקרהו הסתרה והירח לקות והוא אבוד האור לגמרי והנשארים לא זה ולא זה
|
- worship: in thought, in speech, in act???
|
ויש בהם שלוש מפנים אחרים אבל אלו שזכרנו די
|
- qualities of the astrological signs: constant, tropical, bicorporal
|
טבעי המזלות שלשה קיים מתהפך בעל ב' גופות
|
- qualities of the elements:
|
וכן ביסודות שלש
|
- completely light, completely heavy, both light and heavy
|
מהם קל במוחלט מהם כבד במוחלט מהם כבד וקל בהצטרף
|
- completely thick, completely thin, both thick and thin
|
ומצד אחר מהם עב במוחלט ומהם דק במוחלט ומהם עבים ודקים בהצטרף
|
- extremes (hot and dry – cold and moist) and the mediate
|
ומצד אחר מהם קצוות באיך כמו החם והיבש והקר והלח והנשארים מתמצעים בין ב' אלו להשתתפם עם הנשארים בא' מאיכיותיהם
|
|
|
מיני תנועות הגשמים ג'
|
- form the center, to the center, around the center
|
מן האמצע אל האמצע סביב האמצע
|
- straight, rotative, spiral
|
מיני התנועות מצד אחר שלשה ישרה וסבובית ומורכבת משתיהן הנקראת סלזונית
|
|
|
הנה התבאר שהעולמות ג' ושבכל א' מהם מצוי טבע השלוש בדרך שביארנו גם באלוה בתאריו כמו שזכרנו לא זולת זה
|
- souls: vegetative, animal, rational
|
גם בנמצאות מצוי טבע השלוש כי הנפשות ג' במין הצומחת והחיונית והמדברת
|
- plants: tree, grass, vegetable
|
מיני הצומח ג' האילן והעשב והירק והעשב נשאר זמן כמו האילן והירק מזריע ומתיבש בתוך שנה
|
- animals: walking, flying, reptile
|
מיני החי ג' המהלך והמעופף והשח והמדבר לא יחלק לפי שלא יתרבה במין
|
- faculties: natural, animal, rational
|
מיני הכחות ג' טבעי חיוני ונפשיי
|
- dimentions: length, width, depth
|
המרחקים ג' האורך ורחב והעומק
|
- foundations of the three dimentions: line, surface, body
|
ולוקחים התחלותיהם מג' הקו והשטח והגשם
|
- triangular shape – the first of the plaine shapes, built of three lines, the foundation of all polygons, therefore the ancients thought it is an element (Aristotle, On the Heavens, III, 306b3-29)
|
התמונות הישרות הקוים השטוחות הראשונה מג' גבולים והוא המשולש וכל שאר התמונות ישרות הצלעות הרבות אליו יותרו ולזה חשבוהו הראשונים יסוד כמ"ש הפילוסוף בב' מהשמים והעולם
|
- sciences: mathematics, physics, metaphysics
|
החכמות ג' הלמודיות והטבעיות והאלהיות
|
- figures of universal syllogisms (Aristotle, Prior Analytics)
|
תמונות ההקש המולידות ג' כמ"ש בראשון מספר ההקש
|
- analogies: equality, excess, defect
|
מיני ההקשה הראשונים ג' אם שווי אם תוספת אם חסרון
|
- proportions: arithmetical, geometric, harmonic
|
מיני היחס הכוללים ג' המספריי המדותיי הנגוניי
|
- faculty of speech: noun, verb, statement (Aristotle, De Interpretatione, I, 16a1-3)
|
הדבור אצל הפילוסופים שלשה שם ופעל ומלה כמו שהתבאר בתחלת ספר המליצה
|
- perseverance of the elements: not existing and not perishable, permanent and perishable, permanent
|
מיני הטבעים בהתמדה ולא התמדה שלשה לא הוה ולא נפסד באלוה מתמיד במין נפסד באיש כיסודות והמורכב מהם מתמיד באים כמו המלאכים והגלגלים והכוכבים
|
- main leading faculties: generative, growing, nutritive
|
הכחות המנהיגות הראשית ג' מוליד ומגדל וזן
|
- things that are found in the souls: states, faculties, passions (Aristotle, Nicomachean Ethics, II.5, 1105b19-28)
|
הדברים הנמצאים בנפש ג' מקרים כחות ותכונות כמ"ש הפילוסוף בשני מספר המדות
|
- kindes of love: love of goodwill, love of pleasure, love of utility (Aristotle, Nicomachean Ethics, VIII, 2-3, 1155b17-1156a21)
|
מיני האהבה ג': אהבת מעלה אהבת הנאה אהבת תועלת כמ"ש ארסטו בתחלת הח' מס' המדות
|
- parts of the soul: desire (action), sensation, thought (truth) - "what affirmation and negation are in thinking, pursuit and avoidance are in desire" (Aristotle, Nicomachean Ethics, VI.2, 1139a17-31)
|
בנפש ג' חלקים אחד לפועל והוא התאוה ושנים לשפוט והם החוש והשכל ומה שהוא בשכל חיוב ושוללות הוא בתאוה דרישה ובריחה כמ"ש בו' מס' המדות
|
- matters: necessary, impossible, possible
|
החמרים בגזרות ג' מחויב ונמנע ואפשר שהמשולח מטבע האפשר והא מין ממיניו לפי דעת האחרונים
|
- cones: sufficient, supplementary, deficient (Apollonius, The Conical Sections, book I)
|
החתוכים הנופלים במחודד בעגול ג' המספיק הנוסף והחסר כמ"ש במאמר הראשון מספר אבולוניוס בחרוטים
|
- social conducts [types of life]: enjoyment, political, contemplative (Aristotle, Nicomachean Ethics, I.5, 1095b13-1096a5)
|
מיני מנהגי המדינות כפי מה שיראה ארסטו בא' מס' המדות ג' והם התענוג הכבוד העיון
|
|
|
Four
|
הארבעה
|
Properties of the number four
|
|
- the first composite number
|
תחלת מספר מורכב
|
- the first even-times-even number
|
ותחלת זוג הזוג
|
|
|
ותחלת מרובע שיהיה בפועל
|
- 1+2+3+4=10 → the end of the first rank
|
וחבורו משלים י' שהם בפנים מספרי המעלה הראשונה
|
|
|
ושרשו חציו
|
|
|
והוא חצי מעוקבו
|
Tetrad in the existences
|
אמנם בנמצאות איך ימצא רבוע אחר השלוש
|
- things shared by the existents apart from God: imperfect; caused; have multitude; the faculty of each does not spread to the rest (Plato, Timaeus)
|
כבר ביארו אפלטון בס' טימאוס הרוחניי כשאמר שהנמצאות כלם בלתי האלוה אחר הבדלם בישיותהם ישתתפו בארבעה דברים בהיותם בלתי שלמים בהיותם עלולים בהיותם בעלי רבוי בהיות כח כל א' מהם בלתי מתפשט בכל הנשארים
|
- things in the orbs: orb; star; essence; separate mover
|
ובגלגלים ד' דברים הגלגל והכוכב הנפש והמניע הנבדל
|
- natures in the orbs: stars, planets; the two luminaries (Maimonides, Guide II.9)
|
בגלגלים המצויים ד' טבעים המכוכב כוכבי הנבוכה שני המאורות כמו שבי' הר"מ ז"ל פי י"א משני
|
- faculties emanate from the orbs: inanimate; vegetative; animal; rational
|
הכוחות השופעות מן הגלגל ד' הדומם הצומח החי המדבר
|
- skills of analogy: proof; argumentation; refutation; rhetorics
|
המלאכות אשר תעשנה ההקש ד' והן המופת והנצוח וההטעמה וההלצה
|
- seasons: cold; hot; summer; winter
|
התקופות ארבעה קור וחום קיץ וחורף
|
- astrological aspects: opposition; triplicity; quartile; sextile
|
מיני המבטים אשר כפיהם יתמזגו ניצוצות הכוכבים ארבעה נכח שליש רביע שתות
|
- opposites: contradiction; correlation; privation and possession; affirmation and negation
|
המקבילות ארבעה ההפכים המצטרפים ההעדר והקנין החיוב והשוללות
|
- qualities: hot; cold; moist; dry
|
האיכיות ארבעה החום והקור הלחות והיובש
|
|
|
וההמזגות המתילדות מהם ד' כמ"ש החכמים
|
- types of “how”: possetion; meaning; natural faculty and nonnatural faculty; causing and effecting
|
מיני האיך קנין ענין כח טבעי ולא כח טבעי הפעל וההפעלות
|
- phlegms: red; black; white; blood
|
הליחות ד' אדומה שחורה לבנה ודם
|
- causes: matter; form; act; purpose
|
הסבות ד' החומר והצורה והפעל והתכלית
|
- directions: west; east; north; south (Aristotle, ?)
|
הרוחות ד' ימה וקדמה צפונה ונגבה והם מוגבלות כמ"ש הפילוסוף בתחלת השני מס' השמים והעולם כשביאר שלגלגל פאות מוגבלות בטבע
|
|
|
מיני השלמויות ד'
|
- acting powers: attraction; retentive; digestion; repulsion
|
הכוחות העובדות הכח ד' מושך מחזיק מעכל ודוחה
|
- heavenly cardines: ascendant; line of the lower midheaven; setting point; midheaven
|
יתדות הרקיע ד' הצומח וקו התהום והשוקע וחצי השמים
|
- primary mental faculties: intellect; mixing; strength; righteousness
|
כחות הנפש הראשונים ד' והם השכל וההמזגה והחוזק והצדק
|
- trees: those that have waters; those that have resins; those that have oils; those that have milky saps
|
טבעי האילנות ד' בעלי מימות בעלי שרפים בעלי שמנים בעלי חלבים כמ"ש בס' עבודת האדמה
|
- primary organs: brain; heart; liver; testicles
|
האיברים הראשונים ד' מוח לב כבד אשכים
|
- medicine faculties (Ibn Rushd)
|
מיני הכחות המיוחסות לרפואות כפי פעולתם בנו ד' כפי דעת האחרונים ובפרט בן רשד בס' הכליות
|
- constructions of the figure (Aristotle)
|
חבורי התמונה ד' ואין בכל חבורי שאר התמונות טבעי, אלא כי זאת התמונה יש שאמר ארסטו בהקש ממה שת[..]ול מחשבת בני אדם בטבע והיא תנליו בלא מלאכה ותוליד כל מיני הסותרים ושאר התמונות בהפך כל אלו כמו שהתבאר בספר ההקש
|
- questions: simple "if"; complex "if"; what; why (Aristotle, Posterior Analytics, II, 1, 89b23-24)
|
מיני הדרישות ד' אם פשוט אם מורכב ומה ולמה כמ"ש הפילוסוף בתחלת השני מס' המופת
|
- other have said these are: if; what; how; why
|
ואחרים אמרו שהם אם ומה איך ולמה
|
|
|
חיות המרכבה ד'
|
|
|
ומרכבות זכריה ד' והם מורות על עניינים נכבדים זכרו מהם החכמים שלפנינו הרבה
|
|
|
ועדין נשאר הרבה אבל אין בזה הספר מקום לדבר בם וכ"ש שסובלות דברים ארוכים ועמוקים ואם יניח השם לנו ניחד בהם מאמר בפני עצמו
|
|
|
Five
|
החמשה
|
Properties of the number five
|
|
- round number – the units of the powers of five are always five
|
מספר עגול סובב על עצמו וזה שהוא שומר עצמו במרובעו ובמעוקבו
|
|
כי מרובע ה' כ"ה
|
|
ומעוקבו קכ"ה
|
|
ואם יכפל יותר ימצא בו פרט ה'
|
|
|
וזה שהחמשה כנקודה אמצעית בין המספרים הט'
|
- a sum of the first square in potentia and the first square in actu
|
והוא חבור המרובע הראשון בכח עם המרובע הראשון בפעל
|
|
|
ויש בו סגולה נפלאה מעידה שהמספרים ט' לבד והיא שהחמשה מרובעו ומרובע מרובעו ומרובע כפלו כמו מעוקבו וזה שמרובעו כ"ה ומרובע כפלו ק' הרי קכ"ה והוא מעוקב ה'
|
|
|
וכל מספר שלפני ה' ערך מרובע ומרובע כפלו אל מעוקבו כערך אותו המספר אל ה'
|
|
|
וכל מספר שאחר ה' הדבר בהפך והנה נאריך בזה במקומו הראוי לו
|
Pentad in the existences
|
ויש בנמצאות דברים כוללים ירוצו מרוצת הה'
|
- foundations of existence: intellect, form, matter, place, time (Plato, Timaeus)
|
מהם כמ"ש אפלטון בס' תימאוס שהחמשה הם שרשי המציאות והם השכל והצורה והחומר והמקום והזמן
|
- subjects of Geometry: point, line, surface, solid, angle
|
נושאי ההנדסה ה' הנקודה הקו והשטח הגשם והזוית
|
- predicates of the sentence: genus, species, difference, property, accident
|
נשואי הגזרות ה' והם הסוג המין ההבדל הסגולה המקרה
|
- corporeal senses: sight, hearing, smelling, taste, touch
|
ההרגשות הגשמיות ה' הראות והשמע והריח והטעם והמשוש
|
- signs of life in the nativities: the two luminaries, the ascendant, midheaven, lot of beauty
|
סימני החיים במולדות אצל ההוברים ה' והם מקומות שני המאורות והצומח וחצי השמים וגורל היופי
|
|
|
ירידת הכח הגלגליי בעולם השפל תלוי במצבי ה' עגולים והם עגול המזלות ועגול ב' ההפוכים ועגול המבדיל בין הנראה תמיד מהגלגל והעגול המבדיל בין הנסתר תמיד
|
- vowels (Nahmanides / Moses Qimhi, Ibn Ezra)
|
התנועות שיבוטאו בהם התארים הגזרים ה' והם פתח צרי חירק שורק חולם והשאר אינם טבעיות ויקראו בעלי הנקוד בתנועות כמו שיאמר רמב"ן בקצורו לנקוד ואבן עזרא בס' ואלה שמות
|
- geometrical perfect solids (Euclid)
|
התמונות הגשמיות שוות התושבות וימוששו מכל צד הם ה' לבד וכבר התבאר עניינם בי"ג מאיקלידס
|
- final types of analogies between things
|
מיני ההקשה האחרונים בין הדברים שיפול ביניהם הם ה' וכבר התבאר עניינם בשער השני
|
|
|
הכוכבים שהשתתפו בנזורות ורחב בלתי קיים הם ה' שצמנ"כ
|
- simple and compound parts of speech: sound, letter, word, section, sentence
|
חלקי הדבור הפשוטים והמורכבים ה' והם הקול האות הגזר התיבה הגזרה
|
- kinds of excellences: three intellectual [and two moral] (Aristotle, Nicomachean Ethics, I.13, 1103a4-13)
|
מיני האשור ה' ג' במחשבי וכו' כמ"ש בסוף הא' מס' המדות
|
- generative faculties of animals: gives birth to its like in its body; lays eggs in its body and [gives birth to] an animal outside its body; lays complete eggs in its body and outside its body; lays incomplete eggs in its body which are completed outside [its body]; generating worms outside its body (Aristotle, Generation of Animals, II.1, 732a25-b7)
|
התולדה בב"ח תמצא על ה' פנים הא' שיוליד בגופו חי כמותו בצורה והב' שיוליד בגופו בצים וחוץ מגופו בע"ח הג' שיוליד בגופו וחוץ מגופו בצים שלמים הד' שיוליד בגופו בצים בלתי שלמים וישלמו בחוץ והה' שיוליד בחוץ תולעים כמ"ש הפילוסוף בי"ו מס' ב"ח
|
| causes of maleness and femalenees: mixture of two, directions, nature of the place, nature of the water and the air (Aristotle, Generation of Animals, IV)
|
סבות הזכרות והנקבות בב"ח ה' והם המזג השנים הרוחות טבע המקום טבע המים והאויר כמ"ש ארסט"ו בי"ח מב"ח
|
|
|
Six
|
הששה
|
Properties of the number six
|
|
- The first even-times-odd number
|
תחלת זוג הנפרד
|
- round number – the units of the powers of six are always six
|
והוא כמו כן מספר כדוריי מתגלגל על עצמו כמו החמשה
|

|
וזה שהששה שומר ג"כ עצמו במרובעו ובמעוקבו כמו החמשה וזה כי ו' על ו' ל"ו
|

|
וו' על ל"ו רי"ו
|

|
ואם יכפל עוד ישמר בכפל ההוא וכן לעולם
|
- the only perfect number among the units
|
והששה מספר שלם ר"ל שחלקיו שוים לכולו לא פחות ולא יותר
|
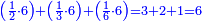
|
וזה שחציו ג' ושלישיתו ב' וששיתו א' הרי ששה
|
|
|
ואין בלעדיו מספר שלם במדרגה הראשונה
|
- the only perfect number among the tens is 28
|
ובשנית ימצא אחד והוא כ"ח
|
- among the hundreds – 496
|
ובשלישית תצ"ו
|
- among the thousands – 81[2]8
|
ובד' שמות אלפים ר"פ ומן הוא והלאה לא ימצא מספר שלם רק בדלוג מדרגות
|
- the perfect numbers are very few; most numbers are either deficient or abundant – analogy to people is added
|
וכל המספרים אם נוספים ואם חסרים וחכמי העיון הוציאו מזה רמז כי השלמים ימצאו מעטים ובפליאה ובדלוג מדינות ודורות
|
- (Aristotle,)
|
ואמנם קראו זה המספר שלם לפי שגדר השלם כמ"ש הפילוסוף הוא אשר אין להוסיף עליו ולא לגרוע ממנו ולזה נק' העגול שלם בתמונות השטוחות והכדור במוגשמות
|
|
|
והאנשים שעניינם כך מעטים
|
|
|
ואמנם רוב האנשים אם חסרים מהראוי להיות בם ואם שיהיו בם דבר לא יתכן שיהיו
|
|
|
ואמנם הדרך בהוצאתם נבארהו כשנגיע אל הסגולות הפרטיות
|
Hexad in the existences
|
וכמה דברים כוללים בנמצאות ירוצו מרוצת הששה
|
- cause of existence: God, intellect, soul, wheel, form, primeval matter (Al-Fārābī)
|
כמ"ש אלפראבי בתחלת ספרו הנק' התחלות הנמצאות שאמר שהתחלות המציאות ששה והם האלוה השכל הנפש הגלגל הצורה ההיולי
|
- directions: upward, down, right, left, forward, backwards
|
הפאות ששה מעלה ומטה ימין ושמאל פנים ואחור
|
|
|
המזלות הצפוניים ו' והם טש"ת סא"ב
|
|
|
והדרומיים ו' מע"ק גד"ד
|
- composition of relations in diverse aspects
|
חבור היחסים הל[קוחי]ם בנושאים מפורדים אמנם הוא בו'
|
|
|
קולות הנגון ו' ובד' מהם מתחיל הטבע האחד מהנעימה והולך עד ששה וכן משם ולמעלה עד לאין תכלית כי מה שאין תכלית לו אי אפשר שיצא אל הפעל לעולם
|
- irrational continuous or discontinuous lines
|
הקוים האלמים מדובקים או נבדלים המתילדים בכל סוג מסוגי הנבדלים הם לעולם ששה ששה
|
- orifices of the human body: eye, ear, nose, mouth, small intestine, lagre intestine (Aristotle, ?)
|
מוצאי המותר בגוף האדם ו' והם העין האזן האף הפה מוצא המותר הדק ומוצא המותר העב ואין ראוי למנות הכפולים רק אחדים כי כמ"ש הפילוסוף לא נכפלו רק מפני היותר טוב
|
- general faculties of the human soul: growth, nourishment, sensation, common sense, imagination, reason
|
כחות הנפש האנושית הכוללים ששה והם צמיחה הזנה הרגש חוש משותף דמיון שכל
|
- joints of the arm, the hand and the finger
|
פרקי הזרוע והיד וכל אצבע ששה וזה שמהכתף עד תחלת היד שנים ומתחלת היד עד ראש כל אצבע א' ובכל אצבע ג' הרי ו' וכלם מתיחסים בהדרגה ביחס מוגבל בטבע אם לא שישנה הטבע על הזרות
|
- joints of the legs to the tip of each toe
|
וכן מתחלת הרגל עד קצות כל אצבעות הרגל
|
- protrusive organs of the face: two eyes, two ears, two nostrils
|
האיברים הבולטים בפנים ו' והם שתי עינים שתי אזנים שתי נחירים ואין ראוי למנות השפתים לפי שהאדם יכול לקפוץ פיו ושפתיו ולא יוכר בם שנוי משאר שטח הפנים
|
- greatest stars in the skys
|
גדלי כוכבי הרקיע כלם יחלקו לו' כמ"ש החכמים
|
- common causes of health and sickness: surrounding air, food and drink, motion and rest, sleeping and waking, emptying and constipation, psychological accidents
|
הסבות המשותפות לבריאות והחולי ששה האויר המקיף מאכל ומשתה תנועה ומנוחה שינה ויקיצה הרקה והסגר חדושים נפשיים
|
|
|
Seven
|
השבעה
|
Properties of the number seven
|
|
- The last prime number among the units
|
הוא מספר ראשון שבמדרגה ראשונה וזה שהמספרים הראשונים שבמדרגה היא בגה"ז
|
- a sum of the first even number and the second odd number (7=2+5)
|
ומספר הז' מורכב מתחלת הזוגות עם שני לנפרדים
|
- a sum of the first odd number and the second even number (7=3+4)
|
ומתחלת הנפרדים עם שני לזוגות ולזה קראוהו קדמוני החכמים מספר כולל
|
- middle between the four composite numbers among the units (4; 6 / 7 / 8; 9)
|
והוא אמצעי בין ארבעת המספרים המורכבים שנים לפניו ושנים לאחריו לפניו ד"ו ואחריו ח"ט
|
|
|
ואם תכפול שבעה יהיו י"ד וזה עולה במחובר מרובעי אב"ג שהם כל טבע המספר כמ"ש למעלה
|
- 1+2+3+4+5+6+7=28 → the only perfect number among the tens
|
וחבור ז' מספר שלם ואין במדרגת העשרות זולתו
|
Heptad in the existences
|
ויש בנמצאות דברים רבים ירוצו מרוצת השבעה
|
|
|
מהם שהכוכבים ז' והם מנהיגי העולם הראשונים ולזה קראום מקדם חכמי ישראל המשרתים
|
- days of the moon’s quadrant
|
ימי כל רבוע מרבועי הירח ז' ובהם יעתקו האוירים והטבעים בבריאות ובחולי
|
|
|
אקלימי הארץ שבעה ואינה חלוקה הנחית אבל נמשכת לכח עליוני כמ"ש חכמי הכוכבים
|
- types of metals: gold, silver, copper, tin, lead, iron, mercury
|
מיני המתכות ז' הזהב הכסף הנחשת הבדיל העופרת הברזל הכסף חי ואע"פ שהברזל לא יותך כפי מה שיחשב הנה איפשר להתיכו בתחבולה נעלמת עד שיותך מהרה כמו העופרת
|
- un talking animals: carnivores, vegetarians, birds of prey, song birds, insects, reptiles, aquatic animals
|
סוגי טבעי הב"ח הבלתי מדברים שבעה חיות טורפות בלתי טורפות עופות דורסים בלתי דורסים שרץ העוף זוחלי עפר
ר"ל שקצים ורמשים חיות המים ותחת כל א' מאלו ישתרגו מינים רבים
|
- sciences: physics, metaphysics, arithmetic, geometry, astronomy, music, politics (Maimonides, and the author of The Book of the Palm [Sefer ha-Tamar])
|
החכמות שיחלקו כפי דעת הפילוסוף ז' והם הטבע והאלהות והמספר וההנדסה וחכמת התכונה הגלגליית וחכמת המוסיקה והחכמה המדינית ויש בחלוקה הזאת חלוף דעות לאחרונים ולא מנו ההגיון לפי שאינו חכמה אבל כלי לבד והאמת כמ"ש הר"ם ז"ל בפ' מ"ג מג' שלשבעה מבוא גדול בעניינים הטבעים והתוריים וכן יאמר בעל ספר התמר סוף ספרו וזה מכלל הדברים הכרתם מחויבת
|
- changes in a human life (The Qanon of Ibn Sinā, Hippocrates)
|
שנויי שנות האדם שבעה כמ"ש בן סינא בראש ספרו בקאנון ואבוקראט בספריו בשביעיות
|
- quatities: line, surface, solid, place, time, number, speech
|
מיני הכמה שבעה הקו השטח הגשם המקום הזמן המספר הדבור
|
- minimal months of existence in which the embryo can survive
|
חדשי עמידת העובר לפחות שיוכל לחיות בו הילוד שבעה וטעם זה ארוך והתבאר היטב בספרי חכמי הכוכבים כי אין בחכמת הטבע די להשלים סבת זה
|
|
|
Nine
|
התשעה
|
Properties of the number nine
|
|
- the first square of an odd number
|
תחלת מרובע מספר נפרד
|
- the sum of its parts equals to the square of the first even number

|
וחלקיו הם כמספר מרובע תחלת זוג לפי שחלקיו שלשה וא' והם ד' שהוא מרובע ב'
|
|
|
ומחוברו כמו הכאתו במספר האמצעי והוא ה'
|
- according to Ibn Ezra, Sefer ha-Shem:
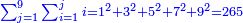
|
וחבור חבורו עולה רס"ה והוא סך מרובעי הנפרדים שבמעלה הא' כמ"ש בן עזרא בס' השם
|
- the last number of the rank of the units
|
ותשעה סוף המדרגה הראשונה מהמספר וזה שהמספרים ט' והאחד עמהם
|
- representation of the products of nine on a circle [Ibn Ezra, Sefer ha-Mispar]
|
והאות ע"ז שאם תעשה עגול ותניח סביבו תשעת המספרים ותתחיל ותכפול ט' על עצמו תמצא המרובע פ"א ותמצא ח' שהוא כנגד פ' אל הימין והא' אל השמאל ואם תכפול ט' על ח' יעלו ע"ב ותמצא ז' שהוא כנגד ע' מימין והב' אל השמאל וכן כל ארבעת מספרים אשר לפני ה' עגול הכלל מימין והפרט משמאל לארבעתם וחמשה לפי שהוא חשבון עגול אמצעי הוא מתגלגל על עצמו והוא בזה הענין כנקודה אמצעית עגול ולזה כאשר תכפול ט' על ד' יעלה ל"ו ותמצא ג' שהוא כנגד ל' עגול אל השמאל וו' שהוא הפרט אל הימין וכן כל הד' שאחר ה' כמו שתראה הנה יתבאר א"כ מזה כי בתשעה טבע הסבוב ולפי שה' באמצע יתחיל ממנו לנטות אל צד אחר מהעגול כי כן משפט מתנועע בסבוב שמנוקדה מהעגול עד חצי העגול ירוץ במצב א' ומשם והלאה מחליף המצב
|
| Special properties of the rank of the units:
|
וכמו שהיות המספרים ט' עם הא' התבאר מצד המספרים עצמם יתבאר מצד מרובעיהם ומצד מעוקביהם
|
- The squares of the units:
|
אמנם מצד מרובעיהם שאם תסדר בטור במספרים הטבעים עד ט' ותניח עליהם או תחתיהם מרובעיהם על הסדר
|
- the units of the squares of the first four numbers are 1; 4; 9[; 6]
|
תמצא שהפרטים ההוים במרובעים עד מרובע ה' חוזרים אחורנית במרובעים שאחריו וזה שהפרטים שאחר שלפני מרובע ה' הם אד"ט
|
- the units of the square of five are five
|
וה' שבאמצע שומר עצמו ואחר חוזרים לאחוריהם כאלו הם הולכים חצי עגול אחר
|
- the units of the next four squares are 6; 9; 4; 1
|
וזה שהפרטים שאחר מרובע ה' הם ו' ט' ד' א'
|
|
|
ואמנם במעוקבים יתבאר הדבר כן אם תסדר מעוקבי המספרים הטבעיים על הסדר עד ט'
|
- the sum of the units of 13 and the units of 93 equals to ten;
|
תמצא פרט המעוקב הראשון עם פרט האחרון הוא כלל והוא ראש המדרגה השנית
|
- the sum of the units of 23 and the units of 83 equals to ten; and so on
|
והשני לא' עם השני לאחרון לפניו עושים כלל וכן תמיד
|
- these sums of the of the units of the first nine cubes represent all the possible ways to divide the number ten into two integers
|
עד האמצעי שהוא ה' שהוא הנקודה לאמצע זה הענין כאלו הוא בחצי קשת העיגול ותמצא בכאן דבר מופלא שכל החלקים השלמין שאפשר שיחלק בם מספר העשרה נמצאים באלו הפרטים פרט לפני ה' עם פרט לאחריו וזה כמו א' וט', ח' וב', ז' וג', ובאמצע שהוא א' מחלקיו בהתחלקו לחצי
|
![\scriptstyle\left[n^2+\left(2n\right)^2\right]:n^3=5:n](/mediawiki/images/math/a/c/d/acd77096f2272cb8b684e8ed0270f8e1.png) . The number where the ratio crosses 1 is 5, so it should be the middle digit. . The number where the ratio crosses 1 is 5, so it should be the middle digit.
|
ומדרך אחרת מצד המעוקב נבאר שהמספרים ט' שכמו שאמרנו במספר ה' שמרובעו ומרובע כפלו שוה אל מעוקבו וכל מספר שלפניו ערך מרובעו ומרובע כפלו אל מעוקבו כערך אותו המספר פשוט אל חמשה ואחר החמשה יתהפך הענין וזה שאז יהיה ערך מרובע המספר ומרובע כפלו אל מעוקבו כערך חמשה אל אותו המספר וזה לאות שהט' שלמות המספר והוא כדמות עגול שלם סובב על עצמו
|
- the units of the first sum
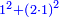 are 5; the second sum are 5; the second sum 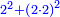 is a product of ten and so on for 1; 2; 3; …; 9 is a product of ten and so on for 1; 2; 3; …; 9
|
וממה שיחזק מה שאמרנו עתה והוא שאם תסדר תשעה המספרים בטור ותשים על כל א' מהם מרובעו ומרובע כפלו תמצא בראשון פרט ה' ובשני כלל וכן עד ט'
|
|
|
וערך כל מרובע מספר מה עם מרובע כפלו אל מרובע אי זה מספר עם מרובע כפלו כערך המספר הפשוט אל המספר הפשוט שנוי בכפל
|
![\scriptstyle\left[n^2+\left(2n\right)^2\right]\sdot\left[m^2+\left(2m\right)^2\right]](/mediawiki/images/math/9/2/e/92ee7ccc084f3dbc9ff4605661fc16be.png) is a square – an algorithm is given for finding this square is a square – an algorithm is given for finding this square
|
ואם תסדר מרובעי המספרים הטבעיים עם מרובעי כפליהם בטור הנה הכאת איזו מדרגה שתהיה מהם עם איזו מדרגה אחרת לעולם מרובע אמנם ידיעת שרשי אלו המרובעים היא ע"ז הדרך תכה הראשון שהוא ה' בשני לו ואחר בג' ואחר בד' וכן ע"ז הסדר תמצא המרובע הראשון שרשו כפל ה' והוא י' ושרש השני יוסיף ה' ושרש ה' יוסיף ה' וכן כלם וזה יקרא הסבוב הראשון ובכל זה הסבוב תמצא המרובעים האחד פרטו והשני כללו וכן לעולם ובסבוב השני והוא שתכה השני מהטור הנז' בכל הבאים אחריו תמצא המרובעים היוצאים ד' דמיוני המרובעים הראשונים ולזה שרשיהם כפלי שרשיהם ולזה הם כלם כללים ובסבוב הג' והוא שתכה הג' בכל הבאים אחריו יהיו המרובעים היוצאים ארבעה דמיוני השניים ולזה שרשיהם כפלי שרשיהם ותמצא האחד כלל והשני פרטו ה' וכן תמיד כדרך הסבוב הראשון וכן החמישי והשביעי והט' סוף דבר הסבובים הזוגות בדרך אחת והנפרדים בדרך אחרת
|
- the units of the first four numbers of the type
![\scriptstyle\left[n+n^2+\left(2n\right)^2\right]](/mediawiki/images/math/6/c/2/6c2974cc18b720735036006b90acc0e1.png) are 6; 2; 8; 4. The fifth number of that type is a product of ten. The units of the next four numbers of this type are again 6; 2; 8; 4 are 6; 2; 8; 4. The fifth number of that type is a product of ten. The units of the next four numbers of this type are again 6; 2; 8; 4
|
וייראה באלו המספרים ר"ל מרובעי המספרים הטבעיים על מרובעי כפליהם שאם תחבר כל א' מהם אל מספרו פשוט תמצא הפרט הראשון ו' עוד ב' עוד ח' עוד ד' והאמצעי שהוא קכ"ה עם מספרו יהיה כלל והמספרים הארבעה שאחריו ה' הענין בם כמו במספרים שלפני ה' וזה אות מופלא שהמספרים ט' לבד
|
| Algorithms for checking if a number is a square or a cube and what are the digits of is its root, considering its units:
|
הנה כבר ביארנו שהמספרים ט' לבד ולזה נקח מהקדמות הנזכרות ראשונה מאזנים למרובעים ולמעוקבים
|
- if its units are 2, 3, or 7 – it cannot be a square
|
וזה שאי אפשר בשום מרובע שיהיה בו פרט ב' או ג' או ז' ואם הוא כן אינו מרובע
|
|
|
|
- if one of its digits is 1– there is 1 or 9 among the digits of its root
|
ואם יש בו א' או ט' היה בשורש
|
- if one of its digits is 4 – there is 2 or 8 among the digits of its root
|
ואם יש בו ד' ב' או ח' היה בשורש
|
- if one of its digits is 6 – there is 4 or 6 among the digits of its root
|
ואם היה בו ו' ד' או ז' היה בשורש
|
- if 5 is its units – there is 5 among the digits of its root
|
ואם בפרט ה' בשורש ה' ג"כ וכן תמיד
|
|
|
ואמנם במעוקבים
|
- if 1 is its unit – there is 1 among the digits of its root
|
אם יש במספר פרט א' הנה במספר בשורש א'
|
- if one of its digits is 2 – there is 2 among the digits of its root
|
ואם יש בו ב' בשורש היה ב'
|
- if one of its digits is 3 – there is 7 among the digits of its root
|
ואם יש בו ג' בשורש היה ז'
|
- if one of its digits is 4 – there is 4 among the digits of its root
|
ואם יש בו ד' בשורש ד'
|
- if one of its digits is 5 – there is 5 among the digits of its root
|
ואם יש בו ה' בשורש ה'
|
- if one of its digits is 6 – there is 6 among the digits of its root
|
ואם ו' בשורש ו'
|
- if one of its digits is 9 – there is 9 among the digits of its root
|
ואם יש בו ט' בשורש ט'
|
| "These are enough proofs that the digits are nine alone"
|
ודי בזה ראיות שהמספרים ט' לבד
|
Ennead in the existences
|
ודע שיש בנמצאות דברים הרבה ירוצו במספרי הט'
|
|
|
מהם כי הרקיעים לא יותר גם בתשיעי ספק לא מעט
|
|
|
השכלים הנפרדים אחר האלוה ית' לפחות ט' וזה כפי דעת הפילוסופי אבל כפי דעת התורה רבו מלמנות
|
- temperaments: one balanced, four simple, four compound
|
המזגים ט' אחד פשוט שוה וארבעה פשוטים וארבעה מורכבים
|
- simple essences: God, intellect, soul, orb, planet, four elements
|
המהויות הפשוטים ט' אלוה השכל הנפש הגלגל הכוכב היסודות הארבעה
|
- agreement and difference between a thing and another: a thing loves a thing; a thing hates a thing; a thing pursues a thing; a thing escapes from a thing; a thing dominates a thing; a thing surrenders to a thing; a thing maintains a thing; a thing damages a thing; a thing alien to a thing (Ikhwān al-Ṣafā)
|
מיני האותות והחילוף שיש בין דבר וזולתו ט' והם טבע יאהב טבע וטבע ישנא טבע, טבע רודף טבע, טבע בורח מטבע, טבע יתגדר על טבע טבע יכנע לטבע, טבע מקיים טבע, טבע מפסיד טבע, והתשעי הוא טבע נכרי לטבע ר"ל שאין ביניהם האותות והתנגדות ואלו הם ט' סוגים יכנסו תחתיהם כל מיני הפעל וההפעלות בעניינים הטבעיים ובס' אכואן אלספא הובאו משלים מפרטי הטבע בכל א' מהם והראשונים היו מונים אלו הסוגים י"ג והאחרונים השיבום אל ט' והנה התבארו דברים אלו בס' השתנות הטבעים
|
- human duplicate organs designed for special actions: eye; ear; nose, lip; teeth; hand; foot; breasts; testicles
|
האיברים שייחדם הטבע במין האנושי חוץ מהגוף לפעולות מיוחדות וכפל אותם הם ט' והם העין האזן האף השפה השניים היד הרגל השדים האשכים
|
- accidents (based on Aristotle, Categories, )
|
סוגי המקרים ט' והם שביארם הפילוסוף בס' המאמרות בהגיון
|
- qualities of the homogeneous bodies that signify differences of form - relying on Aristotle, Meteorology, IV, 8, 383a1-20 - the author explains that Aristotle enumerates 18, but these are actually 9 as Aristotle includes positive properties and their negations, which are not existing things
|
טבעי המתדמי החלקים אשר ספרם הפילוסוף ברביעי מאותות השמים שהם כדמות הבדלים צוריים הם ט' וזה שהוא מנאם י"ח שישובו לט' וזה שהוא מנה הקניינים והעדריהם וההעדרים אינם דברים ישיים ובמקומו יתבאר בבירור
|
- months of human pregnancy (natural scientists, astrologers, Ikhwān al-Ṣafā)
|
חדשי עמידת העובר האנושי בבטן ט' וזה דבר הסכימו בו חכמי הטבע ולא נתנו לזה טעם מספיק אבל האיצטגנינים האריכו בזה בדברים נכונים כמ"ש בספרים הרבה והיותר מספיק בה מה שזכרו מחברי אכואן אלצפא
|
- tastes: sweet; bitter; salty; spicy; sour; acidic; creamy; tasteless – only eight, since astringent should not be included (Ibn Rushd)
|
מיני הטעמים שמנה מתוק מר מליח חריף חמוץ קובץ דשן תפל ואין למנות העפוץ טעם בפני עצמו לפי שהוא אינו אלא תכלית ה[ק]ביצות כמ"ש בן רשד בס' הכליאת
|
|
|
Ten
|
העשרה
|
Properties of the number ten
|
|
- The beginning of the second rank (the tens)
|
תחלת המדרגה השנית והוא כאחד והשני בה עשרים והשלישי שלשים וכן עד צ' ולזה נגזרו לאלו שמות משמות אחד המדרגה הראשונה והפרטים שבין אלו הם מורכבים משתי המדרגות כמו י"ב כ"ג ל"ד מ"ה וכמו שהוא תחלת מדרגה שנית כן המאה תחלת מדרגה שלישית והאלף רביעית וכן תמיד
|
|
|
ואם תחבר המרובעים שיש עד חציו ר"ל תמצאם כמחובר עשרה פשוט
|
|
|
ונהגו ההמון והספרים לגמור בעשרה מפני שהוא כלל וכאלו הביאם הרצון האלהי לזה להורות שהוא סוף הספורים
|
Decade
|
|
- the counted – God; intellect; sphere; star; soul; element; mineral; plant; animal; human
|
וזה שהספורים עשרה האלוה והשכל והגלגל והכוכב והנפש והיסוד והדומם והצומח והחי והמדבר
|
- categories [Aristotle, Categories, 4, 1b]
|
והמאמרות עשרה
|
- commandments (The Book of Creation [Sefer Yetzira])
|
ודברות התורה הקדושה שנמסרו לנו בסיני הם עשרה והם סוד אלהי נכבד בהנהגם בזה המספר וזה הוא שנרמז בס' יצירה עשר ספירות בלי מה
|
- branches of the human tree: ten fingers; ten toes
|
ופארות אילן האדם עשרה למעלה ועשרה למטה והם עשר אצבעות הידים ועשר אצבעות הרגלים
|
| One of the wonders of nature: the counted are following the number - as the units are not larger than 9 or 10, so there is nothing among the universal principles of the existences that is more than 9 or 10, except by a hypothetical division, such as the 12 zodiac signs, or the 28 stations of the moon, that is not a real determined division
|
ומן הפלא הגמור בהמשך הספורים למספר שכמו שהמספר לא יעבור ט' או עשרה כן לא תמצא בכוללי הנמצאות דבר שיעבור זה המספר כי אם בדרך חלוקה הנחית כמו י"ב מזלות וכ"ח מחנות הלבנה וכיוצא באלו שאינה חלוקה מוגבלת יישיית וזה א' מנפלאות הטבע בלא ספק
|
| The author states that he does not elaborate on this since this subject will be discussed in another section of the book dedicated to the nature of existence
|
ולולא יראתיהו מהאריכות ושלא נצא ממה שאנחנו בו הייתי מאריך בביאור עניינים נפלאים גדולים ויקרים על זה הדרוש אבל ייעדנו לו מקום אחר בס' הסכמנו לדבר בו בטבע המציאות
|
| Because of this wonderment and various other those who assumed that the number is a beginning were mistaken
|
ומפני הפליאה הזאת עם אחרות רבות טעו המניחים המספר התחלה
|
| The universal principles mentioned for each number are but a few of many, for the human intellect cannot apprehend them all the more so the distant ones, thus a clear remark on those mentioned is enough
|
ודע שאותם הכוללים שזכרנו בכל מספר ומספר הם מעט מהרבה כי קצרה יד השכל האנושי להשיגם כ"ש לרחוקים מהשלמות ודי הערה גלויה באותם שזכרנו
|
General Properties of Numbers
|
|
Introduction
|
|
| Henceforth some specific qualities of the nature of number will be presented by way of a tale and description
|
ואחר שהגענו לזה המקום נביא קצת סגולות פרטיות מטבע המספר בדרך הגדה וספור
|
| Not as the way used by Euclid in the Elements, books 7-9, because the number does not require this, since the practical counting verifies any hypothetical proposition, even there the reader will not rest until checking it through the counting test, hence you find Euclid at the end of every proposition brings a numerical example, and not just for the numerical propositions, but also for the geometric propositions. Every matter that could be examined with numbers is translated to numbers, as in most of the propositions of the second book of Euclid's Elements
|
לא כדרך שעשה אקלידס בז' ושמיני ותשיעי מספרו כי המספר אינו צריך לזה אחר שהספירה המעשית תאמת כל הקדמה מונחת גם שם לא ינוח לב הקורא עד יבחננו במבחן הספירה וכן תמצא מפרש אוקלידס בסוף פי' כל הקדמה מהם מביא משל מספריי ולא בהקדמות המספריות לבד אבל גם בהנדסיות כל מה שאפשר לבחון הענין במספר יושב אל מספר כמו רוב הקדמות המאמר השני מאקלידס
|
| Some people argue that Euclid needed this as a proposition for a few of the cases of the tenth book of the Elements, but the author claims that he has checked it and did not find it so and he concludes that Euclid's method in books 7-9 is nothing but a rational comprehension that should be rejected
|
וקצת אנשים אמרו שהוצרך אקלידס לזה להיות לו בהקדמה לקצת מקומות מהמאמר העשירי מספרו ואנחנו חפשנו ולא מצאנו הענין כן א"כ דרך אקלידס בשלשת המאמרים הנז' היא נגיעת השכל לא זולת וזה ממה שראוי שירוחק בכל מקום
|
| The author declares that by this he wishes to satisfy "Our lord, the great king, may God grant him success" [which could be a reference to king Robert of Anjou]
|
וכ"ש באשר אנחנו בו להפיס בו דעת אדונינו המלך הגדול יצליחהו ה'
|
| Therefore, narrative propositions are presented below, which could be proven by counting, collected from the predecessors or formulated by the author himself, according to his testimony
|
ולזה נביא ההקדמות ספוריות ותעיד בהם ונלקט מה שמצאנו מזה לאשר קדמונו ומה שחדשנוהו אנחנו
|
| He who adds to this will be granted long life and peace
|
והמוסיף אחרינו שנות חיים ושלום יוסיפו לו
|
A list of arithmetical statements concerning general properties of the numbers – without demonstrations or proofs
|
|
- The Rule of Three: For every three proportional numbers, the product of the first by the third is the same as the product of the mean by itself

|
כל שלשה מספרים מתיחסים הנה הכאת הא' בג' כהכאת האמצעי בעצמו
|
- The Rule of Four: If there are four, the product of the extremes is the same as the product of the means.

|
ואם היו ארבעה תהיה הכאת הקצוות כהכאת האמצעיים
|
- The smallest numbers in a certain proportion divide the numbers that maintain their proportion – the smaller ones to small numbers and the larger ones to large numbers.
|
קטני המספרים על יחס מה הנה הם ימנו המספרים אשר על יחסם הקטן לקטן והרב לרב
|
- Each of the smallest numbers in a certain proportion is relatively prime to the other; and this proposition can be reversed.
- If
 are proportional [and if are proportional [and if  and and  are the two smallest numbers possible in this proportion] then are the two smallest numbers possible in this proportion] then  and and  are prime to each other and vice versa are prime to each other and vice versa
|
קטני המספרים על יחס מה הנה כל א' מהם ראשון אצל האחר וזאת ההקדמה מתהפכת
|
- When there are two numbers, each of which is relatively prime to the other, and each of them is multiplied by itself, then each of the products is relatively prime to the other.
- If a and b are prime to each other then a2 and b2 are prime to each other
|
כאשר היו ב' מספרים כל א' מהם ראשון אצל האחר והוכה כל א' מהם בעצמו הנה כל א' משתי ההכאות ראשון אצל האחר
|
- Likewise, if two [numbers] are relatively prime to two other [numbers], and they are multiplied by each other and the two others are [multiplied] by each other, then the two products are relatively prime to each other.
- If a and b are prime to c and d then a·b is prime to c·d
|
וכן אם היו שנים ראשונים אצל שנים אחרים והוכו זה בזה והשנים האחרים זה בזה הנה שתי ההכאות ראשונות זו לזו
|
- When there are two numbers, each of which is relatively prime to the other, and they are multiplied by each other, then the product is relatively prime to each of the two numbers [sic].
- If a and b are prime to each other then a·b is prime to a and b
|
כאשר היו ב' מספרים כל א' מהם ראשון אצל האחר והוכו זה בזה הנה אותה ההכאה מספר ראשון אצל כל א' משני המספרים
|
- When there are two numbers, each of which is relatively prime to the other, then their sum is relatively prime to each of the two numbers.
- If a and b are prime to each other then a+b is prime to a and b
|
כאשר היו שני מספרים כל אחד מהם ראשון לאחר הנה מקובץ שניהם ראשון אצל כל אחד משני המספרים
|
- When there are as many numbers as they may, successive by ratio, and the extremes are relatively prime to each other, then the smallest numbers of this ratio are relatively prime to each other; and this proposition can be reversed.
- If
 are proportional and are proportional and  and and  are prime to each other then are prime to each other then 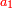 and and  are prime to each other and vice versa. are prime to each other and vice versa.
|
כאשר היו מספרים כמה שיהיו וימשכו על יחס והיו הקצוות ראשונים זה לזה הנה קטני המספרים על אותו היחס וזאת ההקדמה מתהפכת
|
- When there are as many numbers as they may, successive by a certain ratio, and the first does not count the second, then none of them counts the other.
- If
 are proportional and are proportional and 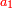 is not a divisor of is not a divisor of  then none of the numbers then none of the numbers  is a divisor of any of the other. is a divisor of any of the other.
|
כאשר היו מספרים כמה שיהיו וימשכו על יחס מה והראשון מהם לא ימנה השני אין מהם מספר ימנה האחר
|
- If the first counts the last, then it count the second.
- If
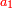 is a divisor of is a divisor of  then then 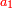 is a divisor of is a divisor of 
|
ואם היה הראשון מונה האחרון הנה הוא מונה השני
|
- When numbers fall between numbers and they follow each other by a certain ratio, then as many numbers that fall between these two numbers, so many fall between every two numbers of the same ratio and all are following by the same ratio.
- If
 are proportional and for a given are proportional and for a given  and and  there are numbers there are numbers 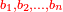 so that so that  are proportional then for every i =1, 2, …, n there are n numbers are proportional then for every i =1, 2, …, n there are n numbers  so that so that  are proportional of the same proportion are proportional of the same proportion
|
כאשר נפלו מספרים בין מספרים וימשכו קצתם לקצתם ביחס מה הנה כסך מה שיפול מן המספרים בין ב' אותם המספרים כן יפול בין כל שני מספרים מאותו היחס וימשכו כלם ביחס א'
|
- When there are two numbers, each of which is relatively prime to the other, and some numbers fall between them that follow [each other] by a certain ratio, then as many numbers that fall between the two of them, so many fall between the first and each of them; and this proposition can be reversed.
- If a and b are prime to each other and
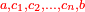 are proportional, then [there are n numbers are proportional, then [there are n numbers 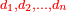 so that so that  are proportional and there are n numbers are proportional and there are n numbers 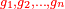 so that so that 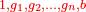 are proportional] and vice versa are proportional] and vice versa
|
כאשר היו ב' מספרים כל א' מהם ראשון אצל האחר ונפלו ביניהם מספרים ונמשכו ביחס מה הנה כסך המספרים שיפלו בין שניהם כן יפלו בין הא' וכל א' מהם וזאת ההקדמה מתהפכת
|
- The ratio of the square numbers to each other is as the ratio of their roots to each other duplicated.
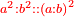
|
המספרים המרובעים יחס קצתם אל קצת כיחס שרשיהם קצתם אל קצת שנוי
|
- When each of the proportional numbers is multiplied by itself, then all the products are also proportional; and if you multiply the products by the original numbers, the resulting products, which are cubes, are also proportional; and so on, if they are further multiplied [the products] are proportional.
- If
 are proportional then are proportional then 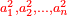 are proportional and are proportional and 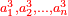 are proportional. are proportional.
|
המספרים המתיחסים כשהוכה כל א' בעצמו הנה כל ההכאות ג"כ מתיחסות ואם תכה ההכאות במספרים הראשונים יהיו כמו כן ההכאות השניות שהם מעוקבים מתיחסים וכן אם יוכו עוד לעולם יתיחסו
|
- If a2 is a divisor of b2 then a is a divisor of b and vice versa
|
כאשר ימנה המרובע מרובע אחר הנה צלעו ימנה צלעו ובהפך
|
- The same is for a3 and b3
|
וכן במעוקב
|
- If a and b are prime to each other then there is no number c so that a:b=b:c
|
כל שני מספרים שהאחד מהם ראשון אצל האחר אין יחס הראשון אל השני כיחס השני אל מספר אחר
|
- then

- and vice versa
|
כאשר היו ב' מספרים שטחים מתדמים ל ר"ל ששני צלעות המספר האחד השטוח על יחס שני צלעות המספר השטוח האחר הנה יפול ביניהם מספר יתמצע ביחס ואותו האמצעי נולד מקטן צלע א' מהשטחים עם גדול צלע האחר וזאת ההקדמה מתהפכת
|
|
|
כאשר היו ב' מספר מוגשמים מתדמים הנה יפלו ביניהם ב' מספרים וימשכו ארבעתם ביחס והוצאת אלו השנים בשתכה תחלה קטן שתי צלעות א' מהמוגשמים בשני מהמוגשם האחר והיוצא תכהו בצלע הגדול מכל א' מהמוגשמים והשניים שיצאו הם האמצעיים וזאת ההקדמה ג"כ מתהפכת
|
- If
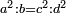 then b is a square number then b is a square number
|
כאשר היו ב' מספרים והיה יחס א' מהם אל האחר כיחס מספר מרובע אל מספר מרובע והיה א' מרובע הנה האחר מרובע
|
- If
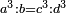 then b is a cubic number then b is a cubic number
|
ואם היו ביחס מספר מעוקב אל מספר מעוקב והיה הא' מעוקב הנה האחר מעוקב
|
- then
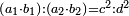
|
המספרים השטוחים המתדמים יחס א' אל האחר כיחס מספר מרובע אל מספר מרובע
|
- then
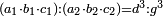
|
והמוגשמים המתדמים יחס א' מהם אל האחר כיחס מספר מעוקב אל מספר מעוקב
|
- then
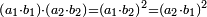
|
המספרים השטוחים המתדמים כשיוכו זה בזה יתקבץ מההכאה מספר מרובע ושרשו הכאת קטן צלע מא' מהם בגדול האחר
|
- then
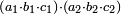 is a cubic number is a cubic number
- and its root is a product of the root of
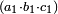 by the root of by the root of 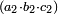
|
המספרים המוגשמים המתדמים כשיוכו זה בזה יתקבץ מספר מעוקב ושרשו שתכה שורש א' מהמוגשמים בשורש האחר והיוצא הוא השרש המבוקש ואמנם אמרתי השרש לפי שהוא מספר נגדר לעולם
|
- If
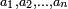 are proportional, and are proportional, and 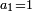
- then for every i=1, 2, …, n:
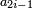 is a square and is a square and 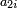 is a cube '''perhaps add "sic"''' is a cube '''perhaps add "sic"'''
|
כאשר היו מספרים מתיחסים מתחילים מהאחד הנה הג' מרובע והד' מעוקב והחמישי מרובע והששי מעוקב והז' מרובע וכן ימשך לעולם
|
- If
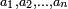 are proportional, are proportional, 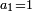 and and 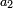 is a square is a square
- then all the numbers
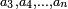 are squares are squares
|
כאשר היו מספרים מתיחסים מתחילים מהא' והיה השני מרובע הנה הנשארים כלם מרובעים
|
- If
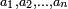 are proportional, are proportional, 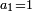 and and 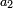 is a cube is a cube
- then all the numbers
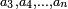 are cubes are cubes
|
ואם היה מעוקב יהיו כולם מעוקבים
|
- If
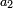 is not a square is not a square
- then none of the numbers
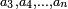 is a square [contradictes the above] is a square [contradictes the above]
|
ואם לא היה השני מרובע אין בהם שום מרובע
|
- If
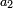 is not a cube is not a cube
- then none of the numbers
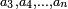 is a cube [contradictes the above] is a cube [contradictes the above]
|
ואם לא היה השני מעוקב אין בהם שום מעוקב
|
|
|
כאשר היו מספרים מתיחסים מתחילים מהאחד הנה כל מספר א' ימנה האחרון מהם הנה הוא ימנה אשר ילוה לאחד
|
- If
 is prime then the divisors of is prime then the divisors of 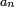 are among the proportional numbers are among the proportional numbers 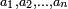 only only
|
ואם היה אשר ילוה לאחד א' הנה לא ימנה הרב מהם כי אם מספר מהם
|
- The smallest number divided by some given primes is not divided by any number other than those given primes
|
כשהיה קטן מספר ימנוהו מספרים ראשונים ידועים הנה לא ימנהו מספר אחר זולתם
|
- If a, b, c are proportional and are the smallest possible numbers in that proportion then a+b is prime to c
|
כאשר היו שלשה מספרים מתיחסים והיו קטני המספרים על אותו היחס הנה כל שנים מהם מחוברים ראשונים אצל הנשאר
|
- If a and b are prime to each other then there is no other number c so that a:b=b:c
|
כל שני מספרים ראשונים זה אל זה הנה אין יחס הראשון אל השני כיחס השני אל מספר אחר
|
- If
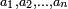 are proportional; are proportional;  and and 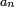 are primes to each other then are primes to each other then 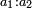 is not equal to is not equal to 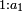 ?? ??
|
כאשר היו מספרים ימשכו קצתם לקצת ביחס מה והיו הקצוות ראשונים זה לזה הנה אין שעור הראשון אצל השני כשעור האחד אל המספר האחר
|
- If
 are proportional then are proportional then 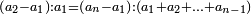
|
כאשר היו מספרים נמשכים אל יחס מה וחוסר מכל א' מהשני והאחרון כמו הראשון הנה שעור מה שישאר מהשני אצל הראשון כשעור מה שישאר מהאחרון אצל כל המספרים אשר לפניו כאשר יקובצו
|
- If 2a-1 is prime to b then 2a-1 is prime to 2b
|
כל מספר נפרד ראשון אצל מספר אחר הנה הוא ראשון אצל כפלו
|
- If a is prime to b then the divisor of a is prime to b
|
כשהיו שני מספרים ראשונים זה אל זה הנה אשר ימנה א' מהם הוא ראשון לאחר
|
- If p is a prime number and the divisor of a·b then is p a divisor of a or b
|
כל ב' מספרים יוכה א' מהם באחר וימנה אותה ההכאה מספר ראשון הנה אותו המספר הראשון ימנה א' משני המספרים אשר הוכו זה בזה
|
- Proportional numbers are proportional by ??
|
המספרים המתיחסים הנה הם בחילוף ובתמורה ובהבדל ובהרכבה יתיחסו
|
|
|
כשהוכה מספר בשני מספרים הנה יחס שתי ההכאות א' מהן לאחרת כיחס המספר למספר
|
- If a=p·(b·c) is a product of a prime number p and a composite number b·c then p and b·c are divisors of a and so are the divisors of b·c and every number that is a product of p by any divisor of b·c. No number other than those is a divisor of a
|
כל מספר שטוח יהיה א' מצלעותיו מספר ראשון והמספר השני מורכב הנה הוא ימנוהו צלעותיו וכל מספר ימנה צלעו המורכב וכל מספר יתקבץ מהכאת צלעו הראשון בכל מספר ימנה צלעו המורכב ולא ימנהו מספר אחר בלתי אלו
|
- If a=(b·c)·(d·g) is a product of two composite numbers b·c and d·g then b·c and d·g are divisors of a and so are the divisors of b·c and d·g and every number that is a product of b·c by any divisor of d·g or a product of d·g by any divisor of b·c. No number other than those is a divisor of a '''sic?'''
|
כל מספר שטוח צלעותיו מספרים מורכבים הנה ימנוהו צלעותיו וכל מספר ימנה כל א' מצלעותיו וכל מספר יתקבץ מהכאת כל א' מצלעותיו בכל מספר ימנה צלע האחר מהם ולא ימנהו מספר אחר בלתי אלו
|
- If
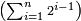 is a prime number then is a prime number then ![\scriptstyle\left[\left(\sum_{i=1}^n 2^{i-1}\right)\sdot2^{n-1}\right]](/mediawiki/images/math/5/2/4/5240941d950fcf6517e4f04e3acc421a.png) is a perfect number is a perfect number
|
כשקובצו מספרים נמשכים על יחס הכפל מן הא' עם האחד והתקבץ מהם כלל והוכה הרב מספר מאותם המספרים במספר א' בלתי השנים הנה אם היה המספר הראשון שוה לכלל אשר קובץ הנה המספר המתקבץ מזה מספר שלם
|
- For a prime number p:
- if
 then then 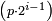 is a superabundant number is a superabundant number
|
ואם היה אותו המספר הראשון פחות מהכלל אשר קובץ הנה הוא מספר נוסף
|
- if
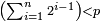 then then 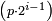 is a deficient number is a deficient number
|
ואם היה המספר הראשון יותר מהכלל אשר קובץ מספר חסר
|
- The abundance / deficiency of
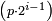 equals to equals to ![\scriptstyle\left[\left(\sum_{i=1}^n 2^{i-1}\right)-p\right]](/mediawiki/images/math/d/a/0/da0afc4f78ef871f81952a2f290c22c4.png) or or ![\scriptstyle\left[p-\left(\sum_{i=1}^n 2^{i-1}\right)\right]](/mediawiki/images/math/2/9/5/2959e7197a06b0b72edbb1040ef5d42f.png)
|
והגעת תוספתו אם היה נוסף וחסרונו אם היה חוסר כמו יתרון מה שבין אותו הכלל אשר קובץ ואותו המספר הראשון
|
- Procedure for finding the perfect numbers:
- The product of an even-times-even number by its corresponding odd number in the table will produce a perfect number, if this odd number is prime.
|
ויש בהוצאת המספר השלם תחבולה א' יותר קצרה והוא שתסדר מספרי זוג הזוג בטור ותניח תחתיו טור הנפרדים הטבעיים מתחיל כנגד ב' מהזוגות הנה כל מספר זוג מהטור העליון שתמצא תחתיו נפרד ראשון ותכהו בו יצא לך מספר שלם ובזה הדרך יצאו המספרים השלימים על סדרם
|
- The unites of the perfect numbers are 6, then 8, then again 6, then 8, and so on
|
ומסגולתם שאם יסודרו אלו השלמים כפי מה שנולדו בטבע תמצא האחד פרטו ה' ואשר אחריו פרטו ח' ואחר ו' ואחר ח' וכן לעולם
|
- For two prime numbers p and q:
|
כאשר קובצו מספרים נמשכים על יחס הכפל מהאחד עמהם והתקבץ מהם כלל והוכה גדול מספר מאותם המספרים במספר שטוח צלעותיו שני מספרים ראשונים בלתי השנים הנה אשר יתקבץ מזה מספר נוסף או מספר חסר
|
- if
![\scriptstyle\left(p\sdot q\right)<\left[\left(\sum_{i=1}^n 2^{i-1}\right)+\left[\left(\sum_{i=1}^n 2^{i-1}\right)\sdot\left(p+q\right)\right]\right]](/mediawiki/images/math/d/2/a/d2afd90cafe829163db22bfa84055049.png) then then ![\scriptstyle\left[\left(p\sdot q\right)\sdot2^{n-1}\right]](/mediawiki/images/math/6/d/a/6da33eb90bcb4511245e22c8c7a87a62.png) is a superabundant number is a superabundant number
|
אמנם אם היה אותו המספר השטוח פחות מהכלל אשר קובץ עם אשר יתקבץ מהכאתם בצלעי אותו המספר השטוח מקובצים הנה המתקבץ מספר נוסף
|
- and its abundance is
![\scriptstyle\left[\left[\left(\sum_{i=1}^n 2^{i-1}\right)+\left[\left(\sum_{i=1}^n 2^{i-1}\right)\sdot\left(p+q\right)\right]\right]-\left(p\sdot q\right)\right]](/mediawiki/images/math/0/5/7/057b6ebf741bca5fb35368372a2da533.png)
|
והגעת תוספתו בהגעת תוספתם על המספר השטוח
|
- if
![\scriptstyle\left(p\sdot q\right)>\left[\left(\sum_{i=1}^n 2^{i-1}\right)+\left[\left(\sum_{i=1}^n 2^{i-1}\right)\sdot\left(p+q\right)\right]\right]](/mediawiki/images/math/a/2/6/a2689c1b254f92dd6a71d7ffd1dd0212.png) then then ![\scriptstyle\left[\left(p\sdot q\right)\sdot2^{n-1}\right]](/mediawiki/images/math/6/d/a/6da33eb90bcb4511245e22c8c7a87a62.png) is a deficient number is a deficient number
|
אמנם אם היה אותו המספר השטוח יותר מהכלל אשר קובץ עם אשר יתקבץ מהכאתו בב' צלעי אותו המספר השטוח מקובצים הנה המספר המתקבץ חסר
|
- and its deficiency is
![\scriptstyle\left[\left(p\sdot q\right)-\left[\left(\sum_{i=1}^n 2^{i-1}\right)+\left[\left(\sum_{i=1}^n 2^{i-1}\right)\sdot\left(p+q\right)\right]\right]\right]](/mediawiki/images/math/e/3/1/e319beb464d016c9118021953702d699.png)
|
והגעת חסרונו בהגעת חסרוניהם מהמספר השטוח
|
|
|
כל ארבעה מספרים נמשכים על יחס הכפל והראשון מהם היותר קטן הנה המשוטח ההוה מהכאת הב' והג' מקובצים בשלישי והד' מקובצים הוא כמו המשוטח ההוה מהכאת המספר הרביעי בראשון והרביעי מקובצים
|
|
|
ואם היה המספר המשוטח ההוה מהכאת הב' והב' מקובצים ברביעי והג' מקובצים כמו המשוטח ההוה מהכאת הרביעי בראשון והרביעי מקובצים הנה המספר המוגשם אשר א' מצלעותיו המספר הג' מהם וצלעו הב' והג' והד' מקובצים וצלעו הב' המספר הב' והג' מקובצים כמו המספר המוגשם אשר א' מצלעותיו המספר הג' מהם והב' המספר הד' מהם והג' המספר הא' והרביעי מקובצים
|
|
|
כל ד' מספרים מתיחסים ביחס הכפל יהיה הא' מהם היותר קטן הנה המספר המשוטח ההווה מהכאת האחרון בראשון והאחרון מקובצים מלבד א' מהם כמו המתקבץ מהכאת המספר הג' מהם במותר מה שבין השטח ההוה מהכאת האחרון בא' והאחרון מקובצים מלבד אחד ובין השטח ההוה מהכאת המספר הג' והרביעי מהם בלתי א' מקובצים בג' והב' בלתי א' מקובצים
|
- [Euclid, Elements, Book II, proposition 4:] For any number divided into [two] parts, whichever they may be, the product of the whole number by itself is equal to the sum of the products of each of the two parts by itself and double the product of one of the two parts by the other.
![\scriptstyle\left(a+b\right)^2=a^2+b^2+\left[2\sdot\left(a\sdot b\right)\right]](/mediawiki/images/math/7/2/7/727b0c35fd93a7a347bed8413905049f.png)
|
כל מספר יחלק בחלקים כמו שיהיו הנה הכאת המספר כלו בעצמו כמו הכאת כל א' משני החלקים בעצמו וכפל הכאת א' משני החלקים באחר כאשר יקובצו
|
- [Euclid, Elements, Book II, proposition 1]

|
כל ב' מספרים יחלק א' מהם בחלקים כמו שיהיו הנה המספר שלא חולק במספר שחולק כמו הכאתו בכל חלקי המספר הנחלק כא[ש]ר יקובצו
|
- [Euclid, Elements, Book II, proposition 5]
![\scriptstyle\left[\frac{1}{2}\sdot\left(a+b\right)\right]^2=\left(a\sdot b\right)+\left[\left[\frac{1}{2}\sdot\left(a+b\right)\right]-a\right]^2](/mediawiki/images/math/1/4/3/1436b388813dc857f5c48fb8c5324880.png)
|
כל מספר זוג יחלק לחציים ולחלקים מתחלפים הנה אשר יהיה מהכאת [חצי] המספר בעצמו כמו ההוה מהכאת החלק הגדול בקטן עם הכאת מותר חצי המספר על החלק הקטן בכמהו
|
- [Euclid, Elements, Book II, proposition 6]
![\scriptstyle\left[\left(\frac{1}{2}\sdot a\right)+b\right]^2=\left[\left(a+b\right)\sdot b\right]+\left(\frac{1}{2}\sdot a\right)^2](/mediawiki/images/math/e/d/0/ed0fccdb234e88ec9c9107530853c90a.png)
|
כל מספר זוג יחלק בשני חציים ויתוסף בו מספר אחר הנה הכאת חצי המספר עם התוספת בכמוהו כהכאת המספר עם התוספת בתוספת והכאת חצי המספר הא' בעצמו
|
- [Euclid, Elements, Book II, proposition 7]
![\scriptstyle\left(a+b\right)^2+a^2=\left[2\sdot\left(a+b\right)\sdot a\right]+b^2](/mediawiki/images/math/5/1/3/513fc9b907ee1cdc2dbe9bb9216406d8.png)
|
כל מספר יחלק בב' חלקים הנה הכאת המספר בכמהו וא' משני החלקים בכמהו כמו ההוה מהכאת המספר בחלק המוכה בכמהו ב"פ והחלק הב' בכמהו
|
- [Euclid, Elements, Book II, proposition 8]
![\scriptstyle\left[\left(a+b\right)+a\right]^2=\left[4\sdot\left(a+b\right)\sdot a\right]+b^2](/mediawiki/images/math/b/3/c/b3ce94dd6ae4e7321478474fa48c51c4.png)
|
כל מספר יחלק בב' חלקים הנה הכאת המספר בכמהו ונוספ עליו כמו א' משני החלקים הנה הכאת המספר עם התוספת בכמהו כמו הכאת בתוספת ארבעה פעמים והכאת החלק האחר בכמוהו
|
- [Euclid, Elements, Book II, proposition 9]
![\scriptstyle a^2+b^2=\left[2\sdot\left[\frac{1}{2}\sdot\left(a+b\right)\right]^2\right]+\left[2\sdot\left[\left[\frac{1}{2}\sdot\left(a+b\right)\right]-a\right]^2\right]](/mediawiki/images/math/4/8/a/48a4cafc5ca8e51bff6710b50982d914.png)
|
כל מספר זוג יחלק בב' חלקים חצאים ובב' מתחלפים הנה כל א' משני החלקים המתחלפים בכמהו כהכאת חצי המספר בכמהו ב' פעמים והכאת מותר חצי המספר על החלק הקטן בכמהו ב"פ
|
- [Euclid, Elements, Book II, proposition 10]
![\scriptstyle\left[2\sdot\left(\frac{1}{2}\sdot a\right)^2\right]+\left[2\sdot\left[\left(\frac{1}{2}\sdot a\right)+b\right]^2\right]=\left(a+b\right)^2+b^2](/mediawiki/images/math/b/f/e/bfe4fce12dd0a607e54ce3db87dd5b6e.png)
|
כל מספר זוג יחלק לחצאים ונוסף בו מספר אחר הנה ההוה מהכאת חצי המספר בכמהו ב"פ והכאת חצי המספר עם התוספת בכמהו ב"פ כהכאת המספר עם התוספת בכמהו והתוספת בכמהו
|
|
|
כל מספר יחלק בשני חלקים מתחלפים ובב' חלקים אחרים מתחלפים הנה הכאת קטן הקטנים בגדול הגדולים ותספת הכאת מותר מה שבין הקטנים במותר מה שבין שני הקטנים והמספר כולו כמו הכאת רב הקטנים בקטן הגדולים
|
- For two numbers which share a common factor, when subtracting repeatedly the smaller from the larger until the remainder is less than the smaller, the remainder will be the greatest common factor of the two given numbers
|
כשהיו ב' מספרים משותפים מתחלפים והובדלו מהגדול דמיוני הקטן עד שישאר פחות ממנו או הוא עצמו וכן בסור הקטן מהגדול עד שיכלה אל מספר הנה הוא גדול משותף בין ב' מספרים
|
- If p and q are primes, then the smallest number which is divided by both of them is p·q
|
אם רצינו למצוא קטן מספר ימנוהו ב' מספרים ידועים אם היו המספרים ראשונים נכה האחד באחר ויגיע דרושינו
|
- For a·c and b·c with a,b relatively prime the smallest number which is divided by both of them is (a·c)·b=(b·c)·a
|
ואם היו משותפים נקח גדול מספר משותפ ביניהם ונקח מספר האחדים שהוא מונה הקטן ושהוא מונה הגדול ותכה הקטן מאלו בגדול המספרים המשותפים או הגדול בקטן המספרים המשותפים כי הכל א' ואותו המספר הוא המבוקש
|
- Example: finding the smallest number divisible by 2, 3, 4:
 '''This doesn't really reflect the two stage process...''' '''This doesn't really reflect the two stage process...'''
|
כשנרצה למצוא מספר קטן ימנוהו מספרים ידועים כאלו נאמר ב'ג'ד' הנה נקח קטן ימנוהו ב"ג והוא ו' ואם היה ו' ימנוהו בג"ד טוב ואם לא נקח קטן מספר ימנוהו ו' וד' והוא כ"ד והוא הדרוש
|
- Finding the smallest number whose parts are given – the same case as above
|
כשנרצה למצוא קטן מספר בו חלקים ידועים הנה נת' מפני ההקדמה שלפני זאת
|
- finding the smallest numbers in a proportion of some proportional given numbers:
|
כשנרצה למצוא קטני מספרים על יחס מוגבל
|
- if the given proportional numbers are primes, then they are the smallest numbers of that proportion
|
אם היו ראשונים הנה הם קטני המספרים על אותו היחס
|
- if the given proportional numbers have a common divisor
|
ואם היו משותפים הנה נקח גדול מספר ימנם
|
- example: 8, 12, 18
|
וכאלו אלו המספרים הם ח' י"ב י"ח וגדול מספר ימנם ב' ונקח מספר אחדים שימנה ב' ח' ומספר השעור שימנה ב' י"ב וכשעור שימנה י"ח ותמצא דו"ט והם קטני המספרים על אותו היחס
|
- Finding the smallest numbers in a given ratio [?] - 2:4=½; 4:12=⅓; 6:24=¼
|
כשנרצה למצוא קטני מספרים על יחסים ידועים בנושאים מפורדים כמו ב' אל ד' חצי ד' אל י"ב שליש ו' אל כ"ד רביע נבקשהו בנושאים נלוים הנה נקח קטן מספר שיש לו חצי
|
- If a and b are primes, then there is no third number proportional to them
|
נרצה לידע כשהיו ב' מספרים אם ימצא להם מתיחס הנה אם היו ראשונים לא ימצא שלישי על יחסם
|
- If a and b have a common divisor: if a is a divisor of b2 then there is a third number proportional to a and b;
- but if a is not a divisor of b2 then there is no third number proportional to a and b
|
ואם היו משותפים נכה הב' בעצמו ואם ימנהו הראשון הנה ימנה להם ג' מתיחס אחריהם ואם לא לא
|
- For three proportional numbers a<b<c: if a and c are prime to each other, then there is no fourth number proportional to a, b, c
|
ואם היו ג' ונרצה לידע אם יש להם רביעי הנה אם היו הראשון והג' ראשונים זה לזה אין להם רביעי
|
- When a and c have a common divisor: if a is a divisor of b·c then there is a fourth number proportional to a, b, c;
- but if a is not a divisor of b·c then there is no fourth number proportional to a, b, c
|
ואם היו משותפים נכה השני בג' ויצא מספר מה הנה אם ימנהו הראשון ימצא להם מספר מספר רביעי ואם לא לא
|
- Algorithm for finding pairs of amicable numbers:
|
כשנרצה למצוא מספרים נאהבים כמו שנרצה
|
- For
![\scriptstyle\left[\left(\sum_{i=1}^n 2^{i-1}\right)+2^{i-1}\right]](/mediawiki/images/math/9/a/c/9ac83e03f12389934e968e5b9a54f1eb.png) and and ![\scriptstyle\left[\left(\sum_{i=1}^n 2^{i-1}\right)-2^{i-2}\right]](/mediawiki/images/math/4/c/1/4c145b2562fabeba5c835d7f7a7cb107.png) prime numbers prime numbers
![\scriptstyle a=\left[\left[\left(\sum_{i=1}^n 2^{i-1}\right)+2^{i-1}\right]\sdot\left[\left(\sum_{i=1}^n 2^{i-1}\right)-2^{i-2}\right]\sdot2^{n-1}\right]](/mediawiki/images/math/4/f/7/4f7d1b0dbb4f89fc68367d9471459075.png) ![\scriptstyle b=\left[\left[\left[\left(2^n+2^{n-3}\right)\sdot2^n\right]-1\right]\sdot2^{n-1}\right]](/mediawiki/images/math/f/e/d/fed0b2f9e6a8f1b32815d1b942f2f33e.png) - a and b are amicable numbers
|
הנה נניח מספרים נלוים על יחס הכפל מן האחד והאחד עמהם ויקובצו המספרים אשר קודם האחרון והאחד עמהם ונוסף על המקובץ המספר אשר קודם האחרון וחסר מהנוסף עליו המספר אשר ילוה מה שקודם האחרון הנה יהיו המספרים המתחדשי' אחר התוספת והחסרון מספרים ראשונים ואין אחד מהם שנים ואם לא יהיו ראשונים תעבור הלאה עד שיצאו אלו המספרים ראשונים והכה משוטח א' מהם באחר במספר אשר קודם האחרון ושמור מה שיצא והוסף על האחרון המספר הרביעי [או] האחד אם היה הא' רביעי ממנו והנה מה שיתקבץ במספר האחרון וחסר מן היוצ[א] מן ההכאה ויהיה הנשאר מספר ראשון תכה זה המספר הראשון אשר קודם האחרון הנה היוצא מן ההכאה עם המספר השמור ישוה כל א' מהם כל חלקי האחר ואלו המספרים המתילדים מזאת התחלה נקראו נאהבים
|
- The product of even by even is even
|
הכאת זוג במספר זוג הוא זוג
|
- The product of even by odd is [even]
|
הכאת זוג בנפרד נפרד
|
- The product of odd by odd is odd
|
הכאת נפרד בנפרד נפרד
|
|
|
כשיוכה מרובע במרובע היוצא יהיה מרובע ושרשו כפל השרש על השרש
|
|
|
וערך מרובע על מרובע ושרשו היוצא בחלוק השרש הגדול על השרש הקטן
|
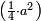 is a square is a square
|
כל מרובע רביעיתו מרובע
|
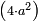 is a square is a square
|
וארבעה דמיוניו מרובע
|
![\scriptstyle\left[n^2-\left[n+\left(n-1\right)\right]\right]](/mediawiki/images/math/5/4/e/54e647dc2cb5d6856381c67937ff7b8f.png) is a square is a square
|
כל מרובע שתחסר ממנו השרש והמספר שלפניו הוא מרובע
|
![\scriptstyle\left[n^2+\left[n+\left(n+1\right)\right]\right]](/mediawiki/images/math/1/6/4/1642161f21eb53b8081872b00544b5ef.png) is a square is a square
|
ואם תוסיף בו השרש והמספר שלאחריו יהיה מרובע
|
|
|
מרחק מרובע ממרובע סמוך לו כמחובר ב' השרשים
|
|
|
כל שני מרובעים סמוכים או רחוקים יוכה שרש א' מהם באחר יגיע מספר מתיחס בין שני המרובעים ההם
|
|
|
אם תסדר החבור הטבעי בטור ותצרף כל מדרגה עם אשר אחריה יתילדו המרובעים
|
|
|
אם תקבץ המספרים עד גבול ותחזור לאחור ותקבץ הכל יעלה כמרובע המספר אשר עמדת בו
|
|
|
אם תסדר המספרים הנפרדים כסדרם והאחד עמהם ותחברם א' א' יתילדו המרובעים הטבעיים
|
- Example: 1; 3; 5; 7; 9; 11
|
כמו שתניח בטור א' ג' ה' ז' ט' י"א
|

|
הנה א' מרובע א'
|

|
תחבר אליו ג' יהיו ד' והוא מרובע ב'
|
|
תחבר אליהם ה' יהיו ט' והוא מרובע ג' וכן תמיד
|
|
|
אם תניח הזוגות הטבעים בטור ותחברם כמו שעשינו בנפרדים יתילדו המרובעים הטבעיים ושרשיהם
|
- Example: 2; 4; 6; 8; 10
|
כמו ב' ד' ו' ח' י'
|

|
הנה ב' א' וצלעו
|
|
נחבר אליו ד' יהיו ו' שהם מרובע ב' וצלעו
|
|
תחבר אליהם ו' יהיו י"ב והוא כמרובע ג' וצלעו וכן לעולם
|
- Forming the cubic numbers using the odds:
|
אם תסדר הנפרדי' הטבעיים בטור כסדרם
|
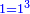
|
הנה הנפרד הראשון והוא א' מעוקב א'
|
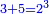
|
וחבור ב' נפרדים אחריו שהם ג' ה' יהיה מעוקב ב'
|
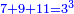
|
ושלשה נפרדים אחר ה' שהם ז' ט' י"א יולידו מעוקב ג'
|
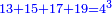
|
וארבעה אחר י"א יולידו המעוקב הרביעי וכן תמיד
|
|
|
ואם תחבר בזה הדרך הזוגות יתילדו המעוקבים כסדרן וצלעותיהם
|
- Forming the odds:
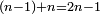
|
אם תסדר המספר הטבעי ותצרף כל מדרגה אל אשר אחריה יתילדו הנפרדים הטבעיים
|
|
|
אם תחבר המעוקבים כסדרם כמו שתרצה והאחד עמהם הנה המקובץ מרובע ושרשו מרובע החבור עד שרש המעוקב שעמדת
|
|
|
חבור המרובעים הנלוים יודע כשתקח מחובר המספר שהוא שורש לאותו המרובע שעמדת בו שמרהו וקח שני שלישי שורש אותו המרובע עם תוספת שלישית אחד ונכפלהו בשמור והעולה הוא מחובר המרובעים עד סוף אותו המספר
|
|
|
חבור המספר פשוט הוא שתכפול איזה מספר שתרצה חבורו בחצי המספר הבא אחריו או בחציו וחצי אחד והעולה הוא המחובר
|
|
|
חבור הנפרדים לבד הוא שתכה המספר האמצעי בעצמו ב' פעמים ותוסיף עליו השרש והוא המבוקש
|
|
|
חבור הזוגות לבד בחשבון תקח חצי סוף החשבון ותכהו בעצמו ותוסיף עליו השרש שלו והוא המבוקש
|
|
|
החבור הטבעי הוא חצי מרובע המספר שעמדנו בו וצלעו
|
- Illustration of the formula:
![\scriptstyle\sum_{i=1}^n i=n\sdot\left[\left(\frac{1}{2}\sdot n\right)+\frac{1}{2}\right]](/mediawiki/images/math/3/0/2/3020018de91a3ca67e97171855f38e62.png) [[I think it's better to write something like Sum_n:n - Sum_{n-1}:n-1=1/2]] [[I think it's better to write something like Sum_n:n - Sum_{n-1}:n-1=1/2]]
|
אם תסדר המספר הטבעי בטור ותשים על כל א' חבורו ותקיש כל א' אל חבורו תמצא כל חבור יוסיף על המספר חצי
|
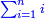 |
36 |
28 |
21 |
15 |
10 |
6 |
3 |
1
|
| n |
8 |
7 |
6 |
5 |
4 |
3 |
2 |
1
|
|
| ל |
כח |
כא |
טו |
י |
ו |
ג |
א
|
| ח |
ז |
ו |
ה |
ד |
ג |
ב |
א
|
|
![\scriptstyle{\color{blue}{1=\left[\left(\frac{1}{2}\sdot1\right)+\frac{1}{2}\right]}}](/mediawiki/images/math/9/6/4/9642ad6342e9ba3d034c4f764ece4387.png) '''I'd put: 1=1x1, 3=(1+1/2)x2, 6=2x3, 10=(2+1/2)x4...''' '''I'd put: 1=1x1, 3=(1+1/2)x2, 6=2x3, 10=(2+1/2)x4...'''
|
דמיון המשל בו שתמצא בכאן א' כמו א'
|
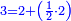
|
וג' כמו ב' וחציו
|

|
וו' ב' דמיוני ג'
|
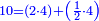
|
וי' שני דמיוני ד' וחציו
|
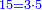
|
וט"ו שלשה דמיוני ה'
|
- The ratio of the bottom line to the upper line is
![\scriptstyle1:n\sdot\left[\left(\frac{1}{2}\sdot n\right)+\frac{1}{2}\right]](/mediawiki/images/math/8/6/8/868269c7b67e057e54f28951727a0e4b.png)
|
וכן תמיד יוסיף בחצי דמיון
|
|
|
כל מספר שתחלקהו בשני חלקים איך שיהיה ותחלק כולו על כל אחד מחלקיו ותכה היוצא מכל אחת משתי החלוקות זה בזה ותשמרהו ואחר תכה אחד משני החלקים באחר ותכהו בשמור יעלה כמרובע המספר
|
|
|
כל חשבון שתקח שלישיתו ותכהו בעצמו ותעלהו מדרגה א' ותחסר ממנו מרובע השלישית יעלה כמרובע המספר
|
|
|
ואם לא היה לו שלישית אבל הוא מוסיף אחד חסר ממנו האחד ותעשה בנשאר כאשר ביארנו והוסף עליו אח"כ המספר שיש לו שלישית והמספר עצמו ויגיע מרובע המספר
|
|
|
ואם יוסיף שנים על שלישית המספר נעשה בהיפך וזה שיוסיף אחד ויהיה מספר שלישי ונעשה כבראשונה ונחסר ממנו בסוף מה שהיינו מוסיפים ויעלה המבוקש
|
|
|
הכאת מעוקב על מעוקב מעוקב ושרשו הכאת שרש א' מהם בשני
|
|
|
חלוק מעוקב על מעוקב מעוקב ואם תחלק שרש הגדול על שרש הקטן תמצא שרשו
|
|
|
אם תסדר המספר הטבעי והא' עמהם ותתחיל ותכה הא' בשני והשני בג' והג' בד' וכן תמיד יתילדו המספרים שהם אמצעיים ביחס בין המרובעים הטבעיים
|
|
|
כל ג' מספרים מתיחסים שתכה שלשתם זה בזה יתקבץ מספר מעוקב ושרשו המספר האמצעי
|
- Every cubic number is between two squares:
|
כל מעוקב יש מצדדיו שני מרובעים
|
![\scriptstyle\left[\left[\left(\frac{1}{2}\sdot n\right)-\frac{1}{2}\right]\sdot n\right]^2<n^3<\left[\left[\left(\frac{1}{2}\sdot n\right)+\frac{1}{2}\right]\sdot n\right]^2](/mediawiki/images/math/b/3/5/b35360945372e8a2345150e6fc0b1fd8.png)
|
אם תחסר מחצי שרש המעוקב חצי א' ותכה הנשאר בשרש המעוקב תמצא שורש המרובע הקטן ואם תוסיף על חצי שרש המעוקב חצי א' ותכהו בשרש המעוקב תמצא שרש המרובע הגדול
|
![\scriptstyle\left[\left[\left(\frac{1}{2}\sdot n\right)+\frac{1}{2}\right]\sdot n\right]^2-\left[\left[\left(\frac{1}{2}\sdot n\right)-\frac{1}{2}\right]\sdot n\right]^2=n^3](/mediawiki/images/math/e/4/5/e45a80471b26560f2fec7a25033e35f2.png)
|
ואם תחסר המרובע הקטן ממרובע הגדול תמצא המעוקב
|
|
|
תראה בזה פליאה נשגבה מאד שאם תסדר המרובעים הטבעיים בטור ותבין בהם ענין זאת ההקדמה תמצא שהמעוקב הראשון ההוה מחסרון מרובע ממנו תמצא שאותם השני מרובעים שזה דרכם ביניהם מרובע א' והמעוקב השני שמצדדיו שני מרובעים הנה ביניהם שני מרובעים והמעוקב הג' בין ב' מרובעים ביניהם ג' מרובעים וכן תמיד יוסיף המרחק בא' כמו זאת הצורה
|
- Examples:

|
אם תסדר המספר הטבעי ותשים תחתיו הזוגות הטבעיים על הסדר ותחבר הראשון והוא א' בזוג הראשון והוא ב' יעלה ג' והוא המספר הא' עם מרובעו ומעוקבו
|
![\scriptstyle{\color{blue}{\left[\left(1+2\right)+\left(2\sdot2\right)\right]\sdot2=\left(3+4\right)\sdot2=7\sdot2=14=2+2^2+2^3}}](/mediawiki/images/math/a/6/f/a6fe7adb4eaa7865bde872ed9080f059.png)
|
תחבר אל הג' הזוג הב' והוא ד' יהיו ז' תכם במספר הב' והוא ב' יעלו י"ד והוא המספר השני עם מרובעו ומעוקבו
|
![\scriptstyle{\color{blue}{\left[\left[\left(1+2\right)+\left(2\sdot2\right)\right]+\left(2\sdot3\right)\right]\sdot3=\left(7+6\right)\sdot3=13\sdot3=39=3+3^2+3^3}}](/mediawiki/images/math/2/c/a/2ca70772497c76b37985cfd04180b40c.png)
|
תוסיף על הז' הזוג הג' והוא ו' יהיו י"ג תכהו במספר הג' שהוא י"ג יעלו ל"ט והוא המספר הג' עם מרובעו ומעוקבו וכן לעולם
|
- The squares of the units are found in two ranks: the squares of the numbers 1, 2, 3 are units themselves and the squares of the rest of the units are found in the rank of the tens
|
ונחתום עתה זה החלק בביאור סגלה נפלאה מהמספר והיא שמרובעי המספרים הט' שהם במדרגה הראשונה הם נשלמים בשתי מדרגות ר"ל האחדים והעשרות וזה שבאחדים לא ימצאו רק מג' מספרים והם אב"ג שמרובעיהם אד"ט והנשארים ישלמו בעשרות
|
- The rank of the units is the beginning and the foundation of all the generated numbers, therefore the squares of the units are analogy for the [squares] of the rest of the ranks:
|
ולפי שהמדרגה הא' התחלה ויסוד לכל המספרים המתחדשים היו מרובעיה דוגמא ומשל לכל המדרגות שאחריה לאין תכלית
|
- The squares in odd ranks – follows the squares in the first rank; the squares in even ranks – follows the squares in the second rank
|
ולפי שמרובעי אחדי המדרגה הראשונה לוקחים משתי המדרגות שהם אחדים עשרות תחזור השלישית אל הראשונה והרביעית לשנית והחמישית לראשונה והששית לשנית וכן תמיד המדרגות הנפרדות מהראשונה והזוגות מהשנית
|
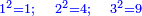
|
וזה לך הפי' במדרגה הראשונה אד"ט מרובעים
|
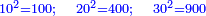
|
ובשלישית מאה ד' מאות ט' מאות ג"כ מרובעים ושרשיהם דמיון שרשיהם אלא שיעלו מדרגה א' כי שרשי אד"ט אב"ג ושרשי אלו יהיו יכ"ל
|
|
|
ואין במדרגה הג' מרובעים ראשי כללים רק אלה כאשר אין באחדים רק אד"ט
|
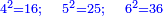
|
ושלמות מרובעי שאר המספרים הטבעיים הם י"ו כ"ה ל"ו וכו'
|
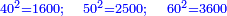
|
וכן במדרגה הרביעית אלף ות"ר אלפים ות"ק ג' אלפים ות"ר ושרשיהם דמיוני שרשי מרובעי האחדים אלא שיעלו מדרגה א' ויהיו ארבעים חמשים ששים וזה שומר הסדר בכל המדרגות עד אין קץ
|
- Since the cubes have three dimensions, the cubes of the units are [found in three ranks] and therefore the cubes of the rest of the ranks are acting as the cubes of the units in these three ranks, in intervals of three ranks
|
ודע שכמו שיש נמשלים במרובעים כן יש במעוקבים ומרובעי ט' המספרים ישלמו בב' מדרגות ר"ל באחדים והעשרות ולזה ידלגו משתים לב' עד לאין תכלית ואמנם המעוקבים הפליא בם הטבע וזה לפי שהמרובע הוא משני מרחקים ישלמו נמשליו בשתי מדרגות ולפי שהמעוקב הוא בעל ג' רחקים ישלמו נמשליו בג' מדרגות וידלגו אח"כ מג' לג' מדרגות עד לאין תכלית כאשר היה דולג במרובעים משנים לשנים
|
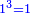
|
וזה שאחד מעוקב אחד ושרשו א'
|

|
כן אלף שהוא רביעי לו מעוקב ושרשו עשרה שהוא אחד שעלה מדרגה
|
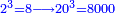
|
וכן שמנת אלפים מעוקב שהוא כנגד שמנה ושרשו עשרים שהוא כנגד שנים ומשם והלאה תשמור הסדר שזכרנו לך
|
- This property is, according to the author, a profound proof that the numbers are nine alone
|
ומכאן ראיה חזקה שהמספרים ט' לבד
|
Epilogue of the surviving section
|
|
| As the numerical properties are endless and therefore further emphasizing concerning them is a waste of time, what is brought is enough for us now, for our intention and according to what was ordered upon us by the great king [again could be a reference to king Robert of Anjou], our lord, may he live and last for long in quiet and safe
|
ולפי שהסגולות המספריות כמעט שאין להם תכלית ולזה ההפלגה בם אבוד הזמן די לנו עתה במה שהבאנו לפי ומה שנצטוינו מהמלך הגדול אדונינו שיחיה ויאריך ימים בהשקט ובטחה
|
| Furthermore, we do not want to attach to this a technical section on actualization of calculations and questions, as much was written about it by all nations due to their need of it in their social affairs, hence it was agreed to conclude here our talk on this first section
|
ולא רצינו לחבר אל זה חלק מלא לפי שחובר על זה הרבה אצל כל האומות לצרכם אליו בענייניהם המדיניים ולזה הסכמנו שיהיה בכאן סוף דברינו בזה החלק הראשון בר"ד
|
| colophon of MS Kepah 36
|
נשלמה העתקת ספרי הראב"ע ז"ל באלול הרמ"ג בקצ"ד לשטרי הצעיר יחיא בן סלי' אלקאפח יצ"ו
|
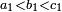 and
and 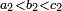 if
if 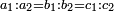

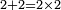 ], i.e. that the multitude of the first effect is not absolute multitude, but only in comparison to a multitude of essences, therefore it is similar to the nature of the number two, whose product does not exceed its sum, unlike all the numbers that follow it, whose products exceed their sums [for every
], i.e. that the multitude of the first effect is not absolute multitude, but only in comparison to a multitude of essences, therefore it is similar to the nature of the number two, whose product does not exceed its sum, unlike all the numbers that follow it, whose products exceed their sums [for every  :
:  ]
] ]
]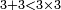 ]
]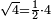

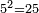
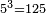
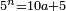
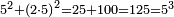
![\scriptstyle\left[n^2+\left(2\sdot n\right)^2\right]:n^3=5:n](/mediawiki/images/math/b/6/9/b6919abe1ff65a25c6f9c44374f4e50c.png)



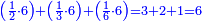

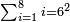
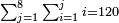 the sum of the divisors of 120 equals its double
the sum of the divisors of 120 equals its double 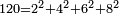
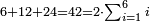

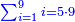
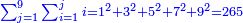
![\scriptstyle\left[n^2+\left(2n\right)^2\right]:n^3=5:n](/mediawiki/images/math/a/c/d/acd77096f2272cb8b684e8ed0270f8e1.png) . The number where the ratio crosses 1 is 5, so it should be the middle digit.
. The number where the ratio crosses 1 is 5, so it should be the middle digit. 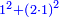 are 5; the second sum
are 5; the second sum 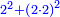 is a product of ten and so on for 1; 2; 3; …; 9
is a product of ten and so on for 1; 2; 3; …; 9 ![\scriptstyle\left[n^2+\left(2n\right)^2\right]:\left[m^2+\left(2m\right)^2\right]=n^2:m^2](/mediawiki/images/math/b/3/6/b362a41c64bc8f1227d1260fa51bb055.png)
![\scriptstyle\left[n^2+\left(2n\right)^2\right]\sdot\left[m^2+\left(2m\right)^2\right]](/mediawiki/images/math/9/2/e/92ee7ccc084f3dbc9ff4605661fc16be.png) is a square – an algorithm is given for finding this square
is a square – an algorithm is given for finding this square![\scriptstyle\left[n+n^2+\left(2n\right)^2\right]](/mediawiki/images/math/6/c/2/6c2974cc18b720735036006b90acc0e1.png) are 6; 2; 8; 4. The fifth number of that type is a product of ten. The units of the next four numbers of this type are again 6; 2; 8; 4
are 6; 2; 8; 4. The fifth number of that type is a product of ten. The units of the next four numbers of this type are again 6; 2; 8; 4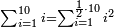


 are proportional [and if
are proportional [and if 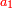 and
and  are the two smallest numbers possible in this proportion] then
are the two smallest numbers possible in this proportion] then  are prime to each other then
are prime to each other then  and
and  there are numbers
there are numbers 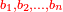 so that
so that  are proportional then for every i =1, 2, …, n there are n numbers
are proportional then for every i =1, 2, …, n there are n numbers  so that
so that  are proportional of the same proportion
are proportional of the same proportion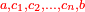 are proportional, then [there are n numbers
are proportional, then [there are n numbers 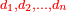 so that
so that  are proportional and there are n numbers
are proportional and there are n numbers 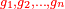 so that
so that 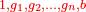 are proportional] and vice versa
are proportional] and vice versa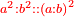
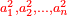 are proportional and
are proportional and 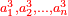 are proportional.
are proportional.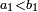 and
and 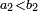 if
if 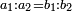

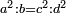 then b is a square number
then b is a square number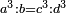 then b is a cubic number
then b is a cubic number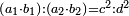
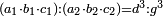
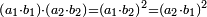
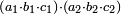 is a cubic number
is a cubic number by the root of
by the root of 
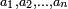 are proportional, and
are proportional, and 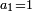
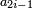 is a square and
is a square and 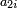 is a cube
is a cube 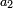 is a square
is a square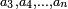 are squares
are squares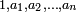 are proportional and b is a
are proportional and b is a 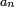 then b is a divisor of
then b is a divisor of 
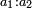 is not equal to
is not equal to 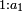 ??
??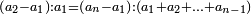
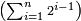 is a prime number then
is a prime number then ![\scriptstyle\left[\left(\sum_{i=1}^n 2^{i-1}\right)\sdot2^{n-1}\right]](/mediawiki/images/math/5/2/4/5240941d950fcf6517e4f04e3acc421a.png) is a perfect number
is a perfect number then
then 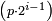 is a superabundant number
is a superabundant number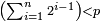 then
then ![\scriptstyle\left[\left(\sum_{i=1}^n 2^{i-1}\right)-p\right]](/mediawiki/images/math/d/a/0/da0afc4f78ef871f81952a2f290c22c4.png) or
or ![\scriptstyle\left[p-\left(\sum_{i=1}^n 2^{i-1}\right)\right]](/mediawiki/images/math/2/9/5/2959e7197a06b0b72edbb1040ef5d42f.png)
![\scriptstyle\left(p\sdot q\right)<\left[\left(\sum_{i=1}^n 2^{i-1}\right)+\left[\left(\sum_{i=1}^n 2^{i-1}\right)\sdot\left(p+q\right)\right]\right]](/mediawiki/images/math/d/2/a/d2afd90cafe829163db22bfa84055049.png) then
then ![\scriptstyle\left[\left(p\sdot q\right)\sdot2^{n-1}\right]](/mediawiki/images/math/6/d/a/6da33eb90bcb4511245e22c8c7a87a62.png) is a superabundant number
is a superabundant number![\scriptstyle\left[\left[\left(\sum_{i=1}^n 2^{i-1}\right)+\left[\left(\sum_{i=1}^n 2^{i-1}\right)\sdot\left(p+q\right)\right]\right]-\left(p\sdot q\right)\right]](/mediawiki/images/math/0/5/7/057b6ebf741bca5fb35368372a2da533.png)
![\scriptstyle\left(p\sdot q\right)>\left[\left(\sum_{i=1}^n 2^{i-1}\right)+\left[\left(\sum_{i=1}^n 2^{i-1}\right)\sdot\left(p+q\right)\right]\right]](/mediawiki/images/math/a/2/6/a2689c1b254f92dd6a71d7ffd1dd0212.png) then
then ![\scriptstyle\left[\left(p\sdot q\right)-\left[\left(\sum_{i=1}^n 2^{i-1}\right)+\left[\left(\sum_{i=1}^n 2^{i-1}\right)\sdot\left(p+q\right)\right]\right]\right]](/mediawiki/images/math/e/3/1/e319beb464d016c9118021953702d699.png)
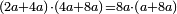
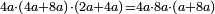
![\scriptstyle8a\sdot\left[\left(a+8a\right)-1\right]=4a\sdot\left[\left[8a\sdot\left[\left(a+8a\right)-1\right]\right]-\left[\left[\left(4a+8a\right)-1\right]\sdot\left[\left(4a+2a\right)-1\right]\right]\right]](/mediawiki/images/math/6/b/c/6bc9bbf210b59b06d2d5ab9c72b105ab.png)
![\scriptstyle\left(a+b\right)^2=a^2+b^2+\left[2\sdot\left(a\sdot b\right)\right]](/mediawiki/images/math/7/2/7/727b0c35fd93a7a347bed8413905049f.png)

![\scriptstyle\left[\frac{1}{2}\sdot\left(a+b\right)\right]^2=\left(a\sdot b\right)+\left[\left[\frac{1}{2}\sdot\left(a+b\right)\right]-a\right]^2](/mediawiki/images/math/1/4/3/1436b388813dc857f5c48fb8c5324880.png)
![\scriptstyle\left[\left(\frac{1}{2}\sdot a\right)+b\right]^2=\left[\left(a+b\right)\sdot b\right]+\left(\frac{1}{2}\sdot a\right)^2](/mediawiki/images/math/e/d/0/ed0fccdb234e88ec9c9107530853c90a.png)
![\scriptstyle\left(a+b\right)^2+a^2=\left[2\sdot\left(a+b\right)\sdot a\right]+b^2](/mediawiki/images/math/5/1/3/513fc9b907ee1cdc2dbe9bb9216406d8.png)
![\scriptstyle\left[\left(a+b\right)+a\right]^2=\left[4\sdot\left(a+b\right)\sdot a\right]+b^2](/mediawiki/images/math/b/3/c/b3ce94dd6ae4e7321478474fa48c51c4.png)
![\scriptstyle a^2+b^2=\left[2\sdot\left[\frac{1}{2}\sdot\left(a+b\right)\right]^2\right]+\left[2\sdot\left[\left[\frac{1}{2}\sdot\left(a+b\right)\right]-a\right]^2\right]](/mediawiki/images/math/4/8/a/48a4cafc5ca8e51bff6710b50982d914.png)
![\scriptstyle\left[2\sdot\left(\frac{1}{2}\sdot a\right)^2\right]+\left[2\sdot\left[\left(\frac{1}{2}\sdot a\right)+b\right]^2\right]=\left(a+b\right)^2+b^2](/mediawiki/images/math/b/f/e/bfe4fce12dd0a607e54ce3db87dd5b6e.png)
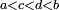 if
if 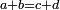 then
then ![\scriptstyle \left(a\sdot b\right)+\left[\left(c-a\right)\sdot\left[\left(a+b\right)-\left(c+a\right)\right]\right]=c\sdot d](/mediawiki/images/math/e/b/3/eb31e9601cbbd0985e9b95ced55be8f5.png)
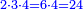
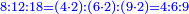
![\scriptstyle\left[\left(\sum_{i=1}^n 2^{i-1}\right)+2^{i-1}\right]](/mediawiki/images/math/9/a/c/9ac83e03f12389934e968e5b9a54f1eb.png) and
and ![\scriptstyle\left[\left(\sum_{i=1}^n 2^{i-1}\right)-2^{i-2}\right]](/mediawiki/images/math/4/c/1/4c145b2562fabeba5c835d7f7a7cb107.png) prime numbers
prime numbers![\scriptstyle a=\left[\left[\left(\sum_{i=1}^n 2^{i-1}\right)+2^{i-1}\right]\sdot\left[\left(\sum_{i=1}^n 2^{i-1}\right)-2^{i-2}\right]\sdot2^{n-1}\right]](/mediawiki/images/math/4/f/7/4f7d1b0dbb4f89fc68367d9471459075.png)
![\scriptstyle b=\left[\left[\left[\left(2^n+2^{n-3}\right)\sdot2^n\right]-1\right]\sdot2^{n-1}\right]](/mediawiki/images/math/f/e/d/fed0b2f9e6a8f1b32815d1b942f2f33e.png)
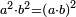
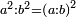
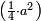 is a square
is a square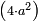 is a square
is a square![\scriptstyle\left[n^2-\left[n+\left(n-1\right)\right]\right]](/mediawiki/images/math/5/4/e/54e647dc2cb5d6856381c67937ff7b8f.png) is a square
is a square![\scriptstyle\left[n^2+\left[n+\left(n+1\right)\right]\right]](/mediawiki/images/math/1/6/4/1642161f21eb53b8081872b00544b5ef.png) is a square
is a square
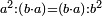
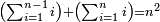
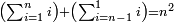

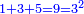


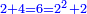
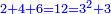
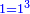
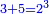
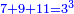
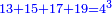
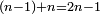
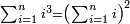
![\scriptstyle\sum_{i=1}^n i^2=\left(\sum_{i=1}^n i\right)\sdot\left[\left(\frac{2}{3}\sdot n\right)+\frac{1}{3}\right]](/mediawiki/images/math/c/e/3/ce3b92c12c7f211d2eccc9b615d868af.png)
![\scriptstyle\sum_{i=1}^n i=n\sdot\left[\frac{1}{2}\sdot\left(n+1\right)\right]=n\sdot\left[\left(\frac{1}{2}\sdot n\right)+\frac{1}{2}\right]](/mediawiki/images/math/b/d/c/bdc2e1765dd2767416cdf4e8c49b5134.png)
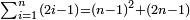
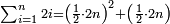
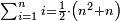
![\scriptstyle\sum_{i=1}^n i=n\sdot\left[\left(\frac{1}{2}\sdot n\right)+\frac{1}{2}\right]](/mediawiki/images/math/3/0/2/3020018de91a3ca67e97171855f38e62.png) [[I think it's better to write something like Sum_n:n - Sum_{n-1}:n-1=1/2]]
[[I think it's better to write something like Sum_n:n - Sum_{n-1}:n-1=1/2]]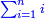
![\scriptstyle{\color{blue}{1=\left[\left(\frac{1}{2}\sdot1\right)+\frac{1}{2}\right]}}](/mediawiki/images/math/9/6/4/9642ad6342e9ba3d034c4f764ece4387.png)
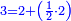

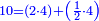
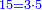
![\scriptstyle1:n\sdot\left[\left(\frac{1}{2}\sdot n\right)+\frac{1}{2}\right]](/mediawiki/images/math/8/6/8/868269c7b67e057e54f28951727a0e4b.png)
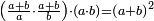
![\scriptstyle a^2=\left[10\sdot\left(\frac{1}{3}\sdot a\right)^2\right]-\left(\frac{1}{3}\sdot a\right)^2](/mediawiki/images/math/6/6/9/669d098149df5c68b5fe9e475df8e3d8.png)
![\scriptstyle\left(a+1\right)^2=\left[\left[10\sdot\left(\frac{1}{3}\sdot a\right)^2\right]-\left(\frac{1}{3}\sdot a\right)^2\right]+a+\left(a+1\right)](/mediawiki/images/math/b/7/3/b738c3c268b17b14598c0d8cc801f193.png)
![\scriptstyle\left(a-1\right)^2=\left[\left[10\sdot\left(\frac{1}{3}\sdot a\right)^2\right]-\left(\frac{1}{3}\sdot a\right)^2\right]-a-\left(a-1\right)](/mediawiki/images/math/0/0/3/00303c8857118de0893cd9a7f7113d6b.png)
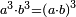
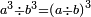
![\scriptstyle n^2:\left[n\sdot\left(n+1\right)\right]=\left[n\sdot\left(n+1\right)\right]:\left(n+1\right)^2](/mediawiki/images/math/2/4/8/2481434f2dedabeea4fe883da3aa7589.png)
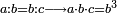
![\scriptstyle\left[\left[\left(\frac{1}{2}\sdot n\right)-\frac{1}{2}\right]\sdot n\right]^2<n^3<\left[\left[\left(\frac{1}{2}\sdot n\right)+\frac{1}{2}\right]\sdot n\right]^2](/mediawiki/images/math/b/3/5/b35360945372e8a2345150e6fc0b1fd8.png)
![\scriptstyle\left[\left[\left(\frac{1}{2}\sdot n\right)+\frac{1}{2}\right]\sdot n\right]^2-\left[\left[\left(\frac{1}{2}\sdot n\right)-\frac{1}{2}\right]\sdot n\right]^2=n^3](/mediawiki/images/math/e/4/5/e45a80471b26560f2fec7a25033e35f2.png)
![\scriptstyle\left[\left[\left(\frac{1}{2}\sdot n\right)-\frac{1}{2}\right]\sdot n\right]^2](/mediawiki/images/math/d/1/c/d1c3f3e39ca11f598ff82ee7157e6a17.png) and
and ![\scriptstyle\left[\left[\left(\frac{1}{2}\sdot n\right)+\frac{1}{2}\right]\sdot n\right]^2](/mediawiki/images/math/8/5/e/85e120d7c925b69eebbf04f11efe4839.png) is rising gradually
is rising gradually ![\scriptstyle\left[1+\left(\sum_{i=1}^n 2i\right)\right]\sdot n=n+n^2+n^3](/mediawiki/images/math/a/f/2/af24ea656570e9534e64304bc5514aa1.png)

![\scriptstyle{\color{blue}{\left[\left(1+2\right)+\left(2\sdot2\right)\right]\sdot2=\left(3+4\right)\sdot2=7\sdot2=14=2+2^2+2^3}}](/mediawiki/images/math/a/6/f/a6fe7adb4eaa7865bde872ed9080f059.png)
![\scriptstyle{\color{blue}{\left[\left[\left(1+2\right)+\left(2\sdot2\right)\right]+\left(2\sdot3\right)\right]\sdot3=\left(7+6\right)\sdot3=13\sdot3=39=3+3^2+3^3}}](/mediawiki/images/math/2/c/a/2ca70772497c76b37985cfd04180b40c.png)