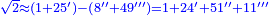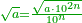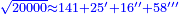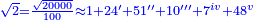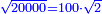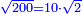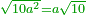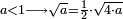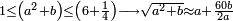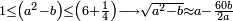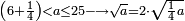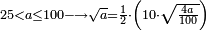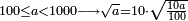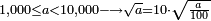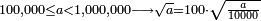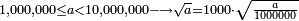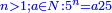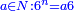Difference between revisions of "Anonymous"
(→Simple fractions to sexagesimal fractions) |
(→Chapter Seven: Conversion of One to the Other) |
||
| Line 7,669: | Line 7,669: | ||
| | | | ||
::Multiply the remainder by sixty and divide by 7; the result is 8, which are seconds and 4 remain. | ::Multiply the remainder by sixty and divide by 7; the result is 8, which are seconds and 4 remain. | ||
| − | |style="text-align:right;"|וערוך הנשאר על ששים וחלק על ז' יצא ח' והם שניים ישאר ד' | + | |style="text-align:right;"|וערוך הנשאר על ששים וחלק על ז' יצא ח' והם שניים ישאר ד‫' |
|- | |- | ||
| | | | ||
| Line 7,676: | Line 7,676: | ||
|- | |- | ||
| colspan="2"| | | colspan="2"| | ||
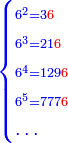
| − | :<math>\scriptstyle{\color{blue}{\frac{2}{7}=\ | + | :<math>\scriptstyle{\color{blue}{\frac{2}{7}=\left(\frac{2\sdot60}{7}\right)^\prime=\left(\frac{120}{7}\right)^\prime=\left(17+\frac{1}{7}\right)^\prime=17^\prime+\left(\frac{1\sdot60}{7}\right)^{\prime\prime}=17^\prime+\left(8+\frac{4}{7}\right)^{\prime\prime}=17^\prime+8^{\prime\prime}+\frac{\frac{4\sdot60}{7}}{60^3}=17^\prime+8^{\prime\prime}+\left(34+\frac{2}{7}\right)^{\prime\prime\prime}}}</math> |
|- | |- | ||
| | | | ||
Revision as of 06:39, 27 December 2022
Contents
[hide]- 1 Introduction
- 1.1 Presentation of the products of units by nine through the arrangement of the nine digits in a circle
- 1.2 One is not a number
- 1.3 One is a number
- 1.4 The Twelve Names that Form Every Number
- 2 Table of Contents
- 3 Interpolated Excerpt
- 4 Chapter One: Addition
- 5 Chapter Two: Subtraction
- 6 Chapter Three: Multiplication
- 6.1 The First Category: [Multiplication of] Integers by Integers
- 6.2 The Second Category: Multiplication of Integers by Fractions
- 6.3 The Third Category: Multiplication of Fractions by Fractions
- 7 Chapter Four: Division
- 8 Chapter Five: Ratios
- 9 Chapter Six: Deducing One from Another
- 10 Chapter Seven: Conversion of One to the Other
- 11 Chapter Eight: Roots
- 12 Notes
- 13 Apparatus
- 14 Appendix I: Glossary of Terms
- 15 Appendix II: Bibliography
| Praise be to the wonderful Almighty God, | [1]תהלה לאל הנעלה הנפלא |
| Who has no beginning or end, | אשר אין לו תחלה ותכלה |
| Who created His world repeating itself, | ויצר עולמו חוזר חלילה |
| Day and night, | יומם ולילה |
| And put wisdom and knowledge in the human heart, | ושם [בלב] האדם חכמה ודעה |
| To calculate a number from one to nine. | לחשב מספר מאחד ועד תשעה |
Introduction |
|
Presentation of the products of units by nine through the arrangement of the nine digits in a circle |
|
| When you write it on a circle you find it recurrent. | גם הוא [כאשר] תכתבנו בעגולה תמצאנו חוזר חלילה |
|
|
|
כי כאשר תערוך תשעה על תשעה יהיה מספר העולה בין שני צדיו האחדים מצד שמאל והעשרות מצד ימין |
|
וכן על ח' ועל ז' ועל ו' |
|
|
|
אך כאשר תגיע לחמשה יתהפכו העשרות לשמאל והאחדים לימין |
|
ועל זה הדרך על ד' ועל ג' ועל ב' |
|
ואם תערכנו על א' יצא לך ט' בעצמו כי כל חשבון הנערך על א' אין לו תוספת |
| If you write nine on the beginning of the circle, you extract them according to this way, only that it will be vice versa to the right and to the left. | ואם תכתוב תשעה בתחלת העגול תוציאם על זה הדרך רק שיתהפך הדבר לימין ולשמאל |
One is not a number |
|
| If the number is like a circle - the one is like a point. | ואם המספר כעגלה האחד כנקדה |
| For, it is the foundation and the cause of all numbers, but it is not a number - it is the essence of a thing. | כי הוא יסוד וסבת כל מספר ואיננו מספר והוא עצם דבר |
| The similar to this are the words of the language that are the secret of every speaker and the source of every reason, but do not utter any reason. | והדומה לזה תבות הלשון שהם סוד כל מדבר ומוצא כל דבר ואינם משמיעים דבר מעניני הדבר |
| From the categories of number it is clear that the one is not a number, for every number is either even or odd, but one is neither. | וממחלקות המספר יתבאר כי האחד איננו מספר כי כל מספר יתחלק לזוג ולנפרד ולא כן האחד |
One is a number |
|
| From another aspect it is also a number. | ומפאה אחרת גם הוא מספר |
General properties of numbers that apply to one |
|
|
ובו נתחבר כל זוג וכל נפרד |
|
ואם חברנו הנפרדים כאשר הם במערכת זה אחר זה יולדו המרובעים והנה האחד עמהם |
|
וכל מספר מחצית שתי פאותיו |
|
והאחד יעשה בפאה אחת מעשה כל מספר בשתים והנו בדרך כל המספר |
|
יסוד כל מספר על שלש דרכים |
|
האחת באחד להיות הנחבר יותר מהנערך |
|
והשנית בשנים להיותם שוים |
|
והשלישית בשלשה להיות הנערך רב מהמחובר |
|
ומשפט כל מספר אחר השלשה בדרך השלשה |
| So, [from this aspect] one is [considered] with the numbers. | והנה האחד עם כל מספר |
Properties of the numbers 2-10 that pertain to one |
|
The number two |
|
| Two is the beginning of the numbers. | ושנים תחלת המספר |
| It is the root of four.
|
והוא שורש ארבעה |
| As the first root of a square number, it represents the properties of the roots: | |
|
וערך הנחבר מעריכת אחד השרשים על האחר אל המרובע הראשון כערך המרובע השני אל הנחבר הנזכר וככה האחד והנו שרש ומרובע |
|
וכל חשבון אם חברנו השרש עם מרובעו יהיה כעריכת השורש על המספר שהוא שני וככה האחד והנו שרש ומרובע |
|
כל שרש שתכפול מרובעו ותוסיף עליו [2]רביעיתו יהיה מרובע ושרשו כמו השרש הראשון מחובר עם חציו וככה האחד |
The number three |
|
| It represents a special property of the proportional triad: | |
|
שלשה מספרים ערך התיכון אל הראשון כערך האחרון אל התיכון אם ערכנו הראשון על האחרון יהיה כמרובע התיכון |
| [ו]אם הראשון כן יהיה האחרון וככה האחד | |
The number four |
|
| Four is a square number | וארבעה מרובע |
| As the first square number, it represents the properties of the square numbers: | |
|
ובין כל מרובע לכפל כפל המרובע אחד שאיננו מרובע וככה האחד |
|
אם חסרנו מרובע ממרובע הקרוב אליו יהיה הנשאר כשנים השרשים הנחברים וככה האחד |
|
כל חשבון אם ערכנו החשבון שהוא לפניו על החשבון שהוא לאחריו ונוסיף אחד יהיה כמרובע החשבון |
|
כל מרובע שתקח שרשו והחשבון שלפניו ותחסרם ממנו ישאר מרובע |
|
ואם תוסיף עליו שרשו והחשבון שלאחריו יהיה מרובע וככה האחד |
The number five |
|
|
החמשה חשבון עגול כי ימצא בכל חשבונו המרובע הראשון |
The number six |
|
|
גם כן ששה רק ששה ימצא בכל חשבונו ולא מרובעו הראשון |
|
והנה האחד ככה |
The number seven |
|
| Holds the following property: | |
|
אם ערכנו שבעה על עצמו ועל כל מספר שלפניו יהיה הנחבר כמרובע כפלו והנה האחד עמהם |
The number eight |
|
| Eight is a cube number [guf šaveh] | השמונה גוף שוה |
| As the first cube number it represents the following properties of the cube numbers: | |
|
ואם חברנו כל חשבון שהוא גוף שוה כאשר הם בתולדת זה אחר זה יהיה מרובע והנה אחד עמהם |
|
ולעולם ימצא גוף שוה בין שנים מרובעים |
|
כשנחסר מחצי קו הגוף חצי אחד ונערוך הנשאר על הקו נמצא שרש המרובע הקטן |
|
וכאשר נוסיף חצי אחד על חצי הקו ונעריכנו על הקו נמצא שרש המרובע הגדול |
|
ואם תחסר מרובע הקטן ממרובע הגדול נמצא הגוף השוה וככה האחד שהוא גוף שוה |
|
ואם תרצה להוציא המרחק שיש בין שני הגופות שהם זה אחר זה קח מרובע קו הגוף הראשון גם מרובע הקו השני וערוך הקו הראשון על השני וחבר הכל אז תמצא המרחק שיש בין שני הגופות וככה האחד |
The number nine |
|
| Nine is a square number. | התשעה חשבון מרובע |
| As the second square it represents the following properties of square numbers: | |
|
והיתרון שיש בין מרובע אחד ובין מרבע אחר לעולם נפרד |
|
ואם תערוך היתרון שיש בין שני המרובעים על שני השרשים כל אחד בפני עצמו |
|
אם הגדול [5]שני לראשון יהיה כמרובע היתרון |
|
| |
|
אם היה שלישי לו תערוך היוצא מעריכת היתרון על שני השרשים על שנים |
|
| |
|
ואם רביעי לו על שלשה וככה האחד |
|
| |
|
אם חלקנו מרובע הקטן על הגדול או הגדול על הקטן לעולם יהיה כמרובע |
|
וכאשר תחלק תשעה על מרובע שבעה יצא בחלוק שביעית ושתי שביעיות שביעית ושרשו ג' שביעיות |
|
והבחינה שתחשב חלקי האחד שבעים יהיה השביעית ושתי שביעיות י"ב ראשונים ס' שניים תשיב הראשונים לשניים ותחבר השניים עמהם יעלה תשע מאות שניים |
|
אם חלקנו מרובע שבעה על תשעה יצא בחלק חמשה וארבע תשיעיות ושרשם שנים ושלישית כי תשעה שחלקנו עליו קם משרש שלשה וככה האחד |
|
אם תרצה לדעת מרובע שרשו ידוע ממרובע ידוע גם שרשו ידוע ערוך המרחק שהוא בין שני השרשים על כל אחד מהשרשים ואשר יהיה תחברם למרובע הידוע אם היה פחות מהמבוקש |
|
או תחסרם אם היה יתר עליו וככה האחד |
| Approximation method for finding a root of a square number | |
|---|---|
| If you want to know the root of a square, integer or fraction, from the preceding square: | אם תרצה לדעת שרש ממרובע שלם או נשבר מהמרובע שלפניו |
|
כמו שרש תשעה[6] [7]ממרובע שנים |
|
חלק המרובע על כפל השרש יצא בחלוק א' ט"ו חברנום עם השרש ויהיה השורש המיוחד ג' ט"ו |
|
רצינו לתקנו ערכנו א' על עצמו ועם ט"ו והנה א"ל וט"ו על ט"ו וכפלנו העולה כי לעולם נכפול השברים וחלקנו הנחבר על ששים והנה ז"ל |
|
| |
|
|
|
וככה תעשה לכל מרובע שתחלוק היתרון שבין שני המרובעים על כפל השרש הראשון ותשמור היוצא הוסיפנו על השרש ויהיה מיושר |
|
|
|
ואחר כן קח היוצא בחלוק וערוך אותו על עצמו ותכפול מרובע השבר ותחלק הכל על כפל השרש המיושר והיוצא תגרע מהשורש המיושר ישאר השרש מהמרובע הגדול |
|
וכן משפט האחד כי המרחק בינו ובין ארבעה שלשה חלקנום על כפל השרש יצא אחד וחצי |
|
לקחנו מרובע היוצא בחלוק עלה שנים ורביע כפלנו הרביע שהם השברים והנה שנים וחצי |
|
| |
|
כל מרובע שתקח שרשו והחשבון שלפני השרש ותחסרם ממנו ישאר מרובע |
|
ואם תוסיף עליו שרשו והחשבון שלאח' אחריו יהיה מרובע |
|
וככה האחד |
The number ten |
|
| Ten is analogous to one [= as a unit in the rank of tens] | ומספר עשרה דומה לאחד |
| As such it represents the following properties: | |
|
|
|
|
וכאשר תרצה לדעת מרובע תשעה תערכנו על אחד שהוא המרחק ותחסרנו מתשעים הדומה לתשעה והנה שמונים ואחד |
|
וכן כל המספר לפי המרחק וככה האחד |
|
ובעבור היות עשרה דומה לאחד נוכל [8]להוציא המרובעים התמימים והנשברים מהערך שיש לו אל עשרה או אל מאה או אל אלף |
|
כמו ע"ה כמה מרובעו |
|
קח ערכו אל מאה והוא ג' רביעיותיו וכמו כן קח ג' רביעיות ע"ה והם י"ח ושלש רביעיות |
|
| |
|
וככה משפט הנשברים עם האחד |
|
וכל מרובע שהוא אחר העשרה יש מערכת המרובע אליו כערך מרובע אחר טרם העשרה אליו |
|
כמו ששה עשר עם ששה ורביע |
|
שערך י"ו אל עשרה כמוהו וג' חמישיותיו |
|
וכאשר תוסיף על ששה ורביע שלש חמישיותיו שהם ג' מה' יעלה עשרה |
|
| |
|
והשרשים יוכיחו כי זה יתחלק על זה כי כשתחלק עשרה על שרש י"ו יעלה שנים וחצי תערכם על עצמם יעלה ו' ורביע וערך עשרה אליו כערך י"ו אל עשרה |
|
וכן אם תקח מהערך עשרה אל י"ו והוא חמש שמניותיו רצינו להוציא חשבון טרם העשרה שיהיה ה' שמיניות עשרה וככה תמצאנו |
|
ערוך י' על ה' יעלה חמשים חלק על שמונה יצא בחלוק ששה נשארו שנים שהם רביע אחד והם חמש שמיניות עשרה |
|
וכאשר נקח ערך י"ו אל י' והוא כמוהו ושלש חמישיותיו ונוסיף על ששה ורביע שלש חמישיותיו יעלה עשרה |
|
| |
|
וכן תמצא לכל חשבון |
|
וכן שרש שבעה על שבעה עם אחד ושלש שביעיות |
|
וככה כלם |
|
גם כן משרש האחד עם המרובעים לפניו ואחריו |
|
וזה יתברר כרביע אחד ששרשו חצי והנה הם כארבעה ושנים |
|
וכן משפט שברי השברים עד אין קץ |
The uniqueness of one – as mean between the proper fractions and the improper fractions |
|
| The proper fractions before one are the inverses to the improper fractions after one | ובעבור היות השרשים בנשברים שהם טרם האחד הפך הנמצאים אחרי האחד |
| Therefore one has the following properties: | |
|
על כן האחד שורש ומרובע וגוף שוה |
|
ידו בכל ויד כל בו[note 1] והוא ראשית הכל ואחרית הכל |
|
ולא יקבל תוספת ומגרעת |
|
ואין לו דמות במספר |
The Twelve Names that Form Every Number |
|
| The names of all numbers are formed by twelve words: | יסוד כל מספר נכלל בשנים עשר שמות |
|
תשעה מהם חוזרים חלילה והם האחדים מאחד ועד תשעה |
|
והשלשה שמות בונים מעלותיו והם העשרות והמאות והאלפים |
|
[9]כי כל חשבון אשר על האלפים חוזרים חלילה על אלו השמות כמו עשרת אלפים ומאת אלף ואלף אלפי אלפים |
Table of Contents |
|
| The chapters representing the eight arithmetical operations applied to all numbers - integers and fractions: | |
| Every number - integer or fraction - is divided into eight chapters and they are: addition of one to another; subtraction of one from another; multiplication of one by another; division of one by another; ratio of one to another; deducing one from another; conversion of one into another; root of one and another. | וכל מספר השלם והנשבר נחלק לשמנה שערים והם מחברת זה עם זה מגרעת זה מזה מערכת זה על זה מחלוקת זה על זה ערך זה אל זה הוצאת זה מזה השבת זה לזה שורש זה וזה |
Interpolated Excerpt |
||||||||||
| שהיה י"ו ישאר ד' | ||||||||||
| If the scales of the remainder we have to subtract from the greater number are greater than the scales of the greater number, we add 9 to the scales of the greater number and then we subtract. | ואלו היה מאזני הנשאר שיש לנו לחסר מהחשבון הגדול יותר ממאזני החשבון הגדול היינו מוסיפים על מאזני החשבון הגדול ט' ואחר כן היינו מחסרים | |||||||||
|
דמיון אחר בנפרדים | |||||||||
|
נאמר החשבון שמנים אלף ושבעת אלפים ושש מאות וחמשים וארבעה והוא מן הנפרדים | |||||||||
|
והדומה לשמנים אלף הוא שמונה באחדים והמרובע הקרוב שעבר הוא ארבעה ושרשו שנים ובעבור היותו [10]במערכת חמישית הנה השרש מאתים | |||||||||
|
ונסיר המרובע שהוא ד' משמונה שהם שמונים אלף אז ישארו לנו [מ"ז] אלף גם תרנ"ד | |||||||||
|
ונכפול השרש ויהיו ת' | |||||||||
| ||||||||||
|
ונחלק מ"ז עליו ולא נוכל לתת לו עשרה ובעבור שיהיו מאה ויש לנו להסיר מרובעו שהוא עשרת אלפים והנה נתן לו ט' שהם צ' ועלה המספר ל"ו אלפים ונשארו לנו י"א אלף גם תרנ"ד | |||||||||
|
והשרש ר"צ | |||||||||
| ||||||||||
|
ויש לנו להסיר מרובע צ' שהוא ח' אלפים ומאה ונשארו ג' אלפים גם תרנ"ד | |||||||||
|
נכפול השרש והנו חמש מאות ושמנים | |||||||||
| ||||||||||
|
||||||||||
| ||||||||||
|
נחלק החשבון הנשאר עליו ויצא ו' | |||||||||
|
הסר מרובע ששה מהנשאר שהיה ע"ד ונשאר ל"ח | |||||||||
|
והנה השרש רצ"ו ומרובעו פ"ז אלפים תרי"ו | |||||||||
|
ומשקל השרש א' חברנו אותו עם משקל ל"ח שהוא הנשאר יותר מהמרובע שהוא שנים עליו שלשה | |||||||||
|
והנה הסתכל משקל החשבון הראשון שהוא הגדול היה שלשה | |||||||||
|
ועוד בקשנו כמה ל"ח שהוא לחסר והנו שנים חסרנוהו ממשקל החשבון הגדול שהיה ג' ונשאר א' | |||||||||
|
וככה ראוי להיות משקל המרובע גם משקל שרשו ערוך על עצמו וככה הוא | |||||||||
| The three algebraic species: root, square, and number | ||||||||||
| All numbers are divided into three categories, which are: root, square, and a number that is neither root nor square. | וכל המספרים הם נפרדים לשלשה ענינים והם שרש ומרובע ומספר שלא יעשה לא שרש ולא מרובע | |||||||||
| You can extract them from each other: | ואתה יכול להוציאם זה מזה | |||||||||
|
כאמרך מרובע שוה לחמשת שרשיו | |||||||||
|
דע כי שרשו ה' ומרובעו כ"ה | |||||||||
|
וכן שלישית מרובע שוה לחמשת שרשיו | |||||||||
|
דע כי שרשו ה' ומרובעו כ"ה | |||||||||
|
וכן שלישית מרובע שוה לד' שרשיו | |||||||||
|
דע כי המרובע יהיה בו י"ב שרשים והמרובע ד' | |||||||||
| The six canonical equations | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| Three simple types of equations | ||||||||||
|
והנה ענין אחד הוא מרובע שוה לשרשים | |||||||||
|
והענין השני מרובע שוה למספר | |||||||||
|
כאמרך ה' מרובעים שוים לפ' | |||||||||
|
דע לך כי המרובע האחד הוא חמישית פ' שהוא י"ו | |||||||||
|
וכן חצי מרובע שוה לי"ח | |||||||||
|
דע כי המרובע הוא ל"ו | |||||||||
|
והענין השלישי שרשים שוים למספר | |||||||||
|
כאמרך ט' שרשים שוים פ"א | |||||||||
|
דע המספר [11]הוא המרובע ושרשו ט' | |||||||||
|
וכן חצי שרש שוה לי' | |||||||||
|
השני עשרים והמרובע מאתים | |||||||||
| You can also find three other composite [types of equations] and they are: | וגם תוכל להוציא מזה שלשה ענינים אחרים מורכבים והם | |||||||||
|
מרובע ושרש שניהם שוים למספר | |||||||||
|
או מרובע ומספר שוים לשרשים | |||||||||
|
או שרשים ומספר שוים למרובע | |||||||||
| The first category:
|
הענין הראשון | |||||||||
|
כאמרך מרובע עם עשרת שרשיו מחוברים עלו ל"ט כמה המרובע וכמה השרש | |||||||||
|
קח חצי השרשים וערכם על עצמם יעלה כ"ה | |||||||||
|
הוסיפם על ל"ט יעלה ס"ד | |||||||||
|
הוצא השרש והוא ח' | |||||||||
|
וחסר מהם חצי השרשים שהוא ה' ישאר ג' והוא השרש המבוקש ומרובע ט' | |||||||||
|
| ||||||||||
|
וכן אם יאמר ב' מרובעים או ג' או יותר עם שרשיו כך וכך שוין למספר כך וכך ככה תעשה | |||||||||
|
קח מרובע אחד וכערכו מן המרובעים קח מן השרשים ומן המספר ועשה כמשפט לחצות ולערוך ולהוסיף ולהוציא השרש ולחסר והנשאר יהיה שרש המרובע האחד | |||||||||
|
וכן אם יאמר חצי מרובע או שלישיתו עם גדריו כך שוים למספר כך ככה תעשה | |||||||||
|
השלם המרובע וכערך שהוספת עליו הוסף על השרשים ועל המספר ועשה כמשפט יצא לך שרש המרובע השלם | |||||||||
| The second category:
|
הענין השני | |||||||||
|
מרובע עם כ"א שוים לעשרת שרשי המרובע | |||||||||
|
קח חצי השרשים וערכם על עצמם יהיה כ"ה | |||||||||
|
הוצא מהם המספר שהוא כ"א ישאר ד' | |||||||||
|
ושרשם ב' | |||||||||
|
אם תגרעהו מחצי השרשים שהוא ה' ישאר ג' והוא שרש המרובע | |||||||||
|
| ||||||||||
|
ואם תוסיפהו על חצי השרשים יעלה ח' והוא כמו כן שרש המרובע | |||||||||
|
כשתחברהו עם כ"א ההם יחד יהיו שוים לי' שרשי המרובע | |||||||||
|
ופעמים שאינו יוצא לשני הפנים האלה | |||||||||
| Know that if the product of half [the number of] the roots is less than the number, then the question is wrong.
|
ודע שאם יהיה היוצא מערך חצי השרשים פחות מן המספר שהשאלה משובשת | |||||||||
| If the product is equal to the number, know that the root of the square is half [the number of] the roots mentioned in the question.
|
ואם יהיה היוצא שוה למספר דע כי שרש המרובע הוא חצי השרשים הנזכרים בשאלה | |||||||||
| The third category
|
הענין השלישי | |||||||||
|
כאמרך ג' שרשים וארבעה שוים למרובע | |||||||||
|
קח חצי השרשים וערכם על עצמם יעלה ב' ורביע | |||||||||
|
[12]הוסיפם על המספר שהוא ד' יהיה ו' ורביע | |||||||||
|
ושרשי ב' וחצי | |||||||||
|
הוסף על חצי השרשים שהם אחד וחצי יעלה ד' והוא שרש המרובע | |||||||||
|
| ||||||||||
| ודע כי לעולם כפל כפל המרובע | ||||||||||
Chapter One: Addition |
השער הראשון במחברת |
|---|---|
| It is divided into two categories: | והוא נחלק לשני ענינים |
|
חבור שלמים עם שלמים |
|
וחבור שברי שברים עם שברים |
The First Category: [Addition of] Integers |
הענין הראשון בשלמים |
Sums |
|
| sum of arithmetic progression of consecutive numbers | |
| When you want to sum numbers increasing by progression as much as you wish. | שתרצה לחבר חשבון הולך וגדל על דרך המספר עד כמה שתרצה |
|
אם החשבון מן הנפרדים |
|
ערוך החשבון שהגיע עדיו על חציו בתוספת חצי אחד והעולה הוא המחובר |
|
והמשל שאלה חברנו האחדים על דרך המספר עד י"א כמה הם |
|
התשובה קח חצי המספר האחרון והוסף עליו חצי אחד והנה ו' ערכם על המספר עלה ס"ו וככה המבוקש |
|
|
|
|
ותדענו שתוסיף על י"א אחד ותערוך העולה על חצי י"א וככה המבוקש |
|
ואם החשבון מן הזוגות |
|
ערוך האחרון על חציו והוסף עליו חצי החשבון |
|
והמשל חברנו מא' עד י' |
|
לקחנו חציו והוא ה' ערכנו אותו על י' והוספנו חמשה שהוא חצי י' ועלה נ"ה וככה המבוקש |
|
| |
|
|
|
|
ותדענו שתוסיף אחד על י' והעולה תערוך במחצית הי' |
| sum of arithmetic progression of consecutive even integers | |
| If you add the numbers [two by two] | ואם תוסיף החשבון בב' |
|
ותתחיל בשנים עד י' פעם |
|
|
|
|
ידוע הוא כי האחרון הוא כ' הוסף עליהם ב' והעולה תערוך על חצי הי' והעולה הוא המבוקש והוא ק"י |
|
|
|
|
או קח חצי [13]המספר האחרון והוא י' הוסף חצי ב' והוא י"א ערכם על י' והעולה הוא המבוקש |
|
| |
|
|
|
|
או קח חצי האחרון והוא ה' הוסיף עליהם חצי אחד והערך על האחרון |
| sum of arithmetic progression of consecutive triples | |
| If you add three by three | ואם תוסיף ג"ג |
|
ותתחיל בג' עד י' פעם |
|
|
|
|
ידוע הוא כי החשבון האחרון הוא ל' הוסף עליהם ג' והעולה תערוך על חצי הי' יעלה קס"ה והוא המבוקש |
|
|
|
|
או קח חצי מספר האחרון והוא ט"ו הוסף עליהם חצי ג' והוא י"ו וחצי ערכם על י' והעולה הוא המבוקש |
|
| |
| sum of arithmetic progression of consecutive quadruples | |
| If [you] add four by four | ואם מוסיף ד"ד |
|
קח חצי רביעיתו |
| sum of arithmetic progression of consecutive quintuples | |
| If five by five | ואם ה"ה |
|
קח חצי חמישיתו |
sum of arithmetic progression of consecutive integers when the first number is equal to the excess 
|
|
|
ולעולם תוסיף חצי אחד והערך על האחרון ודרך אחד לכל התוספות |
This sum of progression is true when adding one by one, starting from 1, or for all excesses, [when the first number is the excess itself] 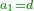
|
וזה חבור המוספים הוא הנכון כשתוסיף א"א תתחיל בא' וכן לכל התוספות תתחיל בחשבונם |
sum of arithmetic progression of consecutive integers when the first number is not equal to the excess 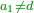
|
|
If you start from 10 and add one by one, or two by two, or three by three, as much as you want, do like this:
|
אך אם תתחיל מי' ותוסיף א"א או ב"ב או ג"ג עד שתרצה ככה תעשה שתוציא לעולם חשבונך מהאחרון כמו שהורתיך למעלה משפט הנפרדים המשפט הזוגות ושמרהו |
|
|
|
ואחר כן אם א"א הוספת עד שהגעת לי"ט והוא מן הנפרדים |
|
וכבר עשית בו משפט הנפרדים אחר כן תפחות א' מן י' וקח מחצית הנשאר וערכם על הי' והעולה תחסר מן השמור שהיה ק"צ והנשאר הוא חשבונך |
|
| |
|
|
|
ואם ב"ב הוספת והיה החשבון האחרון כ' |
|
וכבר הורתיך כמה יעלה והוא ק"י והוא השמור |
|
עכשו חסר ב' מן י' וקח מחצית הנשאר ערכם על מחצית י' והעולה חסר מהשמור והנשאר הוא המחובר מי' עד כ' |
|
|
|
ואם ג"ג הוספת והתחלת בחשבונך מי"ב והיה האחרון ל' אך לא יתכן להתחיל בי' |
|
הוצא חשבונך מן ג' עד האחרון והוא תס"ה ושמרהו |
|
ושוב חסר ג' מי"ב וקח מחצית הנשאר וערכם על שלישית י"ב וחסר העולה מן השמור והנשאר הוא המבוקש |
| Apply this for all the excesses. | וככה תעשה לכל התוספות שתשמר השמות |
| Finding the number of terms in a given sum of multiples | |
| If you want to sum a known number multiplied by the units successively, their [number] is unknown and their sum is so and so, by how many numbers it was multiplied?
|
ואם תרצה לחבר מספר ידוע הספור במספר האחדים על הסדר ואינם ידועים ועלו ככה על כמה מספרים ספר אותו |
|
חלק המספר העולה על המספר שאמר ומה שיצא בחלוק ראה מכמה אחדים יעלה וכמספר האחדים כך [14]מספרים תפש |
|
והמשל ט"ו ערוכים על החשבון על הסדר ועלה המחבר שט"ו על כמה מספרים ספר אותו |
|
חלק שט"ו על ט"ו יצא כ"א |
|
וידענו מהאחדים הספורים מא' ועד ו' הם כ"א |
|
והנה על ששה מספרים ספר אותו והיה המחובר שט"ו |
|
ואם תרצה לדעת כמה המחובר מט"ו ספורים על סדר חשבון מא' ועד ו' |
|
ידענו כי עד ששה יעלה כ"א |
|
וערוך ט"ו על כ"א יעלה שט"ו |
| sum of geometrical progressions of even-times-even numbers | |
| If you want to sum the even-times-even numbers from one to however you wish successively.
|
ואם תרצה לחבר חשבון כפל הכפל מאחד ועד כמה שתרצה על סדר החשבון |
|
|
|
קח מרובע הכפל האמצעי והוא הכפל האחרון אם הכפולים נפרדים וכפול החשבון וחסר ממנו אחד שהוא החלק הראשון והנשאר הוא המחובר |
|
| |
|
והמשל א' ב' ד' |
|
מרובע הב' הוא ד' והוא הכפל האחרון לעולם |
|
ואם הכפולים זוגות |
|
קח מרובע הכפל האמצעי הגדול וחסר ממנו אחד והנשאר הוא המחובר |
|
וכי ירבה ממך דרך[note 2] החשבון שתרצה לדעת מאחד ועד ס"ד כפל הכפל על סדר החשבון ויתקשה עליך להוציא הכפל האמצעי ומרובעו |
|
|
|
ככה תדענו שתתחיל מן השנים וערכם על עצמם והעולה שהוא ד' הוא חשבון שבמדרגה השניה לה וכל החשבון שלפניה כמוה פחות אחד |
|
ושוב וערוך הד' על עצמם יעלה י"ו והוא חשבון שבמדרגה הרביעית לשנית וכל חשבון שלפניה כמוה פחות אחד |
|
ושוב וערוך הי"ו על עצמם יעלה רנ"ו והוא חשבון שבמדרגה השמינית לשניה והחשבון שלפניה כמוה פחות אחד |
|
ושוב וערוך רנ"ו על עצמם יעלה ס"ה אלפים וחמש מאות ושלשים וששה והוא מדרגת י"ו לשניה והחשבון שלפניה כמוה פחות אחד |
|
ושוב וערוך העולה במדרגת י"ו על עצמם ומה שעלה הוא חשבון שבמדרגת ל"ב לשניה והחשבון שלפניה כמוה פחות אחד |
|
ושוב וערוך העולה במדרגת ל"ב ומה שיעלה הוא חשבון שבמדרגת ס"ד והחשבון שלפניה כמוה פחות אחד |
| sum of squares | |
|
|
|
|
ואם תרצה לחבר [15]מרובע אחד ומרובע שנים על דרך החשבון עד מרובע עשרה |
|
ככה תעשה הוסף אחד על עשרה והמחובר תערוך בחצי העשרה יהיו נ"ה ושמרם ואחר כן קח שני שלישי העשרה ותוסיף עליהם שליש אחד יהיה שבעה |
|
| |
|
ואם רצית לחבר מארבעה ואילך עד עשרה |
|
הוצא כל החשבון מאחד ועד עשרה אחר כך חסר האחד מן הארבעה וקח חצי הנשאר וערכהו בארבעה והעולה תערוך בשני שלישי שלשה בתוספת שליש אחד |
|
| |
|
|
|
|
ותדענו שתוציא כל החשבון מא' עד י' ואחר כך הוצא החשבון מא' ועד ג' כל על הדרך שראית והעולה חסר מכל החשבון והנשאר הוא שאלתך |
|
ואם מחמשה ועד עשרה רצית לחבר |
|
הוצא לעולם כל החשבון ואחר כך חסר האחד מן החמשה ומהנשאר קח מחציתם וערכם על החמשה ותערוך העולה בשני שלישי ד' בתוספת שליש אחד לעולם והעולה חסר מן החשבון והנשאר הוא שאלתך |
|
| |
| According to this way for all, keeping the names and the terms. | ועל זה הדרך לכלם שתשמר השמות והמדרגות |
The Second Category: Addition of Fractions |
הענין השני בחבור השברים |
Sexagesimal Fractions |
|
| If you have fractions as the fractions of the astrologers | אם היו בידך שברים כשברי חכמי המזלות |
|
שמחלקים המזל אל שלשים מעלות |
|
והמעלה לששים ראשונים |
|
והראשון לששים שניים |
|
ויהיו ח' מזלות כ"ד מעלה כ"ד ראשונים מ"ד שניים ותרצה לחברם עם ז' מזלות י"ח מעלה מ"ח ראשונים כ"ח שניים |
|
חבר בתחלה השניים והם ע"ב הוסף אחד על הראשונים ישארו י"ב |
|
ושוב חבר הראשונים והם ע"ג הוסף אחד על המעלות ישארו י"ג |
|
ושוב וחבר המעלות והם מ"ג הוסף על אחד על המזלות ישארו י"ג מעלה |
|
ושוב וחבר המזלות והם י"ו הוצא מהם י"ב מזלות שהם חוזרים חלילה |
|
ישארו ד' מזלות י"ג מעלות י"ג ראשונים י"ב שניים |
|
| |
Simple Fractions |
|
| But, the geometricians divide one into a half, a third, a quarter, a fifth, a sixth, a seventh, an eighth, a ninth, and a tenth. | אך חכמי המדות הם מחלקים אחד לחצי ולשליש ולרביע ולחומש ולששית ולשביעית ולשמינית ולתשיעית ולעשירית |
|
ואם תחבר ד' [16]שתותין אל שלשה תשיעיות |
|
בקש חשבון שיהיה לו שתות ותשיעית והוא שתערוך ששה על תשעה יעלה נ"ד והוא המורה |
|
קח ד' שתותין מן המורה והוא ל"ו וחברם אל שלשת תשיעיותיו שהם י"ח יעלה נ"ד והוא אחד שלם |
|
|
|
ותדענו שתדע מאי זה חשבון יצא השתות והוא מששה |
|
קח ד' ששיותיו והוא ד' |
|
וקח ג' תשיעיותיו והוא ב' |
|
חברם וחלקם עליו על ו' יצא אחד |
|
|
|
ותדענו שתדע מאיזה חשבון התשיעית והוא מתשעה |
|
קח ג' תשיעיותיו והוא ג' |
|
וקח ד' ששיותיו והוא ו' |
|
חברם וחלקם על ט' יצא אחד |
|
ואם תחבר ג' שמיניות אל ז' עשיריות |
|
קח המורה והוא שמנים |
|
וג' שמיניותיו הם שלשים חברם אל נ"ו שהם ז' עשיריותיו יעלה ששה ושמונים והוא אחד וששה שמיניות העשירית |
|
| |
|
ואם תחבר ד' שמיניות ושביעית אל ג' ששיות וחמישית |
|
בקש חשבון שיחלק על ארבעת השברים והוא שתערכם זה על זה ויעלה אלף ושש מאות ושמונים והוא המורה |
|
ושמיניתו והוא ר"י |
|
ושביעיתו והוא ר"מ |
|
וששיתו ר"פ |
|
וחמישיתו של"ו |
|
חבר ארבעת שמיניותיו ושביעית שהם אלף ושמנים אל שלשת ששיותיו וחמישית שהם אלף וקע"ו יעלו אלפים רנ"ו והוא אחד וב' שביעיות ורביעית שביעית וחצי רביעית השביעית ועשירית רביעית השביעית |
|
| |
|
ואם תחבר השברים מחצי עד עשירית |
|
בקש חשבון שיהיו לו כל השברים והוא אלפים וחמש מאות ועשרים |
|
וכשתחבר כל השברים יעלה ד' אלפים תתס"א שהם אחד וז' שמיניות וחצי עשירית ושמינית שלישית וחמישית שביעית שמינית |
|
| |
|
ואם תרצה לדעת חשבון שתיתו ורביעיתו היו ה' כמה סך החשבון |
|
הנה המורה הוא כ"ד |
|
קח שתותו ורביעיתו והיו י' חלק עליו המורה יצא שנים וד' עשיריות ערוך היוצא על חמשה יעלה שנים עשר |
|
או ערוך המורה על חמשה והעולה חלק על עשרה [17]עלה שנים עשר |
|
|
|
גם תוכל להוציא מאחד שתקח שתיתו ורביעיתו והוא ב' ששיות וחצי ששית והוא המורה |
|
נחלק עליו אחד יצא שנים ונשאר ששית כלו' נעשה ממנו ששיות ונחלקם בלא שבר על כל ששית וששית |
|
| |
|
ואם תחבר חמישיתו עם שביעיתו ויהיו שנים כמה כל החשבון |
|
ערוך המורה על השנים וחלק על השנים עשר שהם חמשית ושביעית המורה יצא חמשה שלמים וחמש ששיות אחד |
|
או חלק המורה כלו' ל"ה על שנים עשר והיוצא שהוא ג' פחות חצי שתות תערוך על שנים יעלה חמשה וחמש ששיות אחד |
|
|
|
גם תוציאנו מאחד והוא שתקח חמישיתו ושביעיתו ויהיו ב' שביעיות וב' חמישיות השביעית והוא המורה |
|
נחלק עליו אחד יצא בחלוק שנים וישאר שתי שביעיות וחומש שביעית נערכם על שנים והם ארבעה שלמים וד' שביעיות וב' חמישיות השביעית |
|
| |
|
ואם תחבר שלישית רביעית חמישיתו עם שביעית שמינית תשיעיתו ויהיו שנים ותרצה לדעת כמה כל החשבון |
|
בקש חשבון שיהיו לו כל השברים האלה והוא החשבון שיש לו כל החלקים אלפים וחמש מאות ועשרים והוא המורה |
|
ואחר כן קח שלישית רביעית חמישיתו והוא מ"ב וחברהו עם שביעית שמינית תשיעיתו שהוא ה' יהיה הכל מ"ז וחלק עליו המורה יצא חמשים ושלשה וישאר כ"ט |
|
| |
|
או כפול המורה וחלק על מ"ז יצא ק"ז וישאר י"א כמו [18]הענין האחר |
|
| |
|
ובחינתו שתערוך ק"ז על מ"ז ותחבר עם העולה הי"א יהיה הכל חמשת אלפים וארבעים |
|
וכשתקח שלישית רביעית חמישיתו יהיה פ"ד והוא אחד שלם ול"ז חלקים ממ"ז באחד |
|
וכשתקח שביעית שמינית תשיעיתו יהיה עשרה חלקים ממ"ז באחד |
|
חברם עם הל"ז והנה אחד שלם |
Chapter Two: Subtraction |
השער השני במגרעת |
|---|---|
| It is divided into two categories: | והוא נחלק לשני ענינים |
|
מגרעת שלמים משלמים |
|
ומגרעת שברים משברים |
The First Category: [Subtraction] of Integers |
הענין הראשון בשלמים |
| When you wish to know a number that is subtracted repeatedly by the succession of the [natural] numbers, one by one, or two by two, or three by three, from ten to one, the way to extract it is the same as explained for addition, so there is no need to elaborate on that. | שתרצה לדעת חשבון הולך הלוך וחסור על דרך המספר אחד אחד או שנים שנים או שלשה שלשה מעשרה ועד אחד הדרך להוציאו שוה כמו שפרשתי בחבור ואין צורך להאריך בו |
The Second Category: Subtraction of Fractions from Fractions |
הענין השני מגרעת שברים משברים |
Sexagesimal Fractions |
|
| If you have fractions of the astrologers. | ואם שברי חכמי המזלות יהיו בידך |
|
כמו ב' מזלות כ' מעלות מ' ראשונים ומ' שניים ותרצה לגרוע מהם חשבון אחד ולדעת הנשאר כמו א' מזלות י' מעלה מ"ה ראשונים ל' שניים |
|
אתה מתחיל בשניים וישארו י' שניים |
|
ושוב הראשונים מהראשונים ולא תוכל לגרוע הרב מהמעט [..] ומפני זה קח מהמעלות מעלה אחת תהיה ס' ראשונים ותחברם עם הראשונים וגרע מהם מ"ה ראשונים ישארו נ"ה ראשונים |
|
ושוב וגרע המעלות מהמעלות וישארו ט' מעלות |
|
ושוב וגרע מזל אחד מהב' מזלות |
|
ישאר א' ט' נ"ה י' |
|
| |
| If the signs of the subtrahend excceed the minuend signs, you add to them 12 signs, which are the cyclic zodiac signs, then you subtract from the sum and keep the remainder: signs, degrees, minutes and seconds. | ואם מזלות חשבון הנגרעים עודפים על המזלות שאתה גורע ממנו ולא לגרוע אתה מוסיף עליהם י"ב מזלות שהם מזלות הגלגל שהם חוזרים חלילה ומן הנאסף תגרע ותשמור הנשאר בידך מזלות מעלות ראשונים ושניים |
Simple Fractions |
|
| If you have fractions of the geometricians. | ואם שברי חכמי המדות יהיו בידך |
|
כמו שתרצה לגרוע מעשרה ששיתו ורביעיתו ולדעת הנשאר |
|
בקש חשבון שיהיה לו ששית ורביעית והוא י"ב והוא המורה |
|
גרע ממנו ששיתו ורביעיתו ישאר ז' |
|
ודע ערכם [19]והוא חציו וכזה הערך קח מן העשרה והוא חמשה שתותין |
|
או קח מהערך עשרה אל י"ב והוא ה' ששיותיו וקח כזה הערך מן השבעה והוא ה' וה' שתותין |
|
ואם תרצה לגרוע ג' רביעיות אחד מן ד' חומשין ולדעת כמה הנשאר |
|
דע מאיזה חשבון החומש והוא מחמשה |
|
קח ד' חומשין והוא ד' ואחר כך ג' רביעיות החמשה והוא ג' וג' רביעיות תחסרם מן הד' ישאר רביע |
|
ודע מה ערך הרובע מן הה' והוא חצי עשירית |
|
או נקח מן המורה שהוא עשרים ג' רביעיותיו והם ט"ו וגרעם מד' חומשיו שהם י"ו ישאר אחד שהוא חצי עשירית וככה השאר |
|
ואם תרצה לגרוע ארבע חומשיו מן ה' שתותין וג' רביעיות ולדעת הנשאר |
|
בקש חשבון שיהיה לו חומש ושתות והוא שלשים |
|
קח ארבע חמישיות והם כ"ד ותגרעם מחמשה שתותיו ומשלש רביעיותיו שהם מ"ז וחצי יהיה הנשאר כ"ג וחצי |
|
| |
|
ודע ערכם משלשים והוא ז' עשיריות וחצי השתות |
|
ואם תגרע שלישיתו וריביעיתו ונשאר שמונה ותרצה לדעת כמה היה כל החשבון |
|
קח המורה והוא י"ב |
|
וגרע ממנו שלישיתו ורביעיתו ישאר חמשה |
|
חלק עליו המורה יצא ב' וב' חומשין ערכם בשמונה יעלה י"ט וחומש |
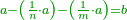
|
|
| According to this way for every remaining number, whether two, or three, or whichever number that remains: extract the denominator, subtract from it the subtracted parts - whether sixths, or fifths, or quarters, or whichever is subtracted - then divide the denominator by the remainder and multiply by the stated remainder.
|
ועל זה הדרך לכל חשבון שישאר שנים או שלשה או איזה חשבון שישאר שתוציא המורה ותגרע ממנו הערכים שגרע הן שתותין או חמישיות או רביעיות או כל מה שגרע ועל הנשאר תחלק המורה והיוצא תערוך בנשאר שאמר |
| Or, divide the stated remainder by what remains from the denominator, after subtracting the subtracted from it, then multiply the result by the denominator. It is the same thing.
|
או חלק הנשאר שאמר על מה שישאר מן המורה כשתוציא ממנו מה שגרע והיוצא תערוך על המורה והדבר שוה |
|
ואם תגרע חמישיתו ומהנותר שביעיתו ונשאר כ"ד כמה היה הכל |
|
דע המורה והוא ל"ה |
|
וערכהו על הנשאר כלומ' כמשפט אשר הזכיר למעלה יצא ל"ה והוא המבוקש |
| This way is correct when the [unknown] number is equal to the denominator like this.
|
וזה הדרך הוא נכון כשיהיו סך החשבון והמורה שוים כמו זה |
|
וכמו אם יקח ל' ויחסר חמישיתו ומן הנותר ששית וישאר עשרים שהחשבון והמורה שוים |
|
| |
| For every number like this, the denominator leads you to the truth. | ובכל חשבון כאלו יוציאך המורה אל האמת |
|
|
|
|
אך אם יקח כ"ה ויגרע חמישיתו ומן הנשאר שביעיתו וישאר י"ז ושביעית שהמורה והחשבון אינם [20]שוים |
|
| |
| For every number such as this if "your eyes shall see thy Teacher and your ears shall hear a word from behind thee... This is the way; walk in it" [Isaiah 30, 20-21]. | ובכל חשבון כיוצא בו אם יהיו עיניך רואות את מוריך אזניך תשמענה דבר מאחריך זה הדרך תלכו בה[note 3] |
|
והדרך לכל חשבון שתבקש חשבון שיהיה לו שביעיות אחרי שתגרע ממנו חמישית |
|
כגון ל"ה שחומשו ז' וישאר כ"ח |
|
| |
|
וישאר כ"ד |
|
ודע מה ערך י"א אל והוא רביעית וה' ששיות רביעית |
|
וכזה הערך קח מי"ז ושביעית שהוא הנשאר והיוצא שהוא ז' ועוד ו' שביעיות |
|
הוסף על י"ז ושביעית יעלה כ"ה והוא מה ששאלת |
| Know it by maintaining the degrees of the fractions and adding one degree to each fraction.
|
ותדענו שתשמור מדרגות השברים ותוסיף על כל שבר ושבר מדרגה אחת |
|
וכן תעשה קח הנשאר והוסף ששיתו שהוא נוסף מדרגה אחת מן השביעית שאמר באחרונה ועל המחובר תוסיף רביעית המחובר שהוא עודף מדרגה אחת על החמישית שאמ' בתחלה והמחובר הוא המבוקש |
|
| |
|
ואם תקח שני חשבונות שאין האחד שוה לחברו ותגרע חמישית האחד משלישית האחר וישארו ששה ותרצה לדעת כמה היה כל אחד ואחד מהחשבונות |
|
בקש חשבון שיהיה לו חמישית ושלישית והוא ט"ו והוא המורה |
|
וקח חמישיתו והוא שלשה והוסף על הששה ויהיה ט' ותערכם על השלשה ויעלה כ"ז וזה הוא החשבון שגרעת ממנו ואחר שידעת זה תוכל להוציא החשבון הנגרע שהוא ט"ו אחרי שחמישיתו שלשה |
| This way is not a general way for every number "and your Teacher shall no longer hide Himself" [Isaiah 30, 20], except when one number is equal to the denominator, such as this and the like, but if none is equal to the denominator it does not apply. | וזה הדרך אינו דרך כלל לכל חשבון ולא יכנף עוד מוריך[note 4] זולתי כשיהיה החשבון האחד שוה למורה כגון זה וכיוצא בו אך אם לא ישוו המורה אל יורה |
|
כגון שהיה האחד י' והשני כ"ד וכשתגרע חמישית עשרה משלישית כ"ד ישאר ששה |
|
ואם תביט אל המורה יוציאך אל כ"ז ואל ט"ו וזה החשבון יודיע אם החשבון האחד ידוע |
|
כמו שיאמר האחד עשרה גרעונו חמישיתו משלישית חשבון שאינו ידוע ונשאר ששה כמה החשבון שאינו ידוע |
|
הדרך בו ולכל חשבון שתוסיף חמישית [21]הידוע על הנשאר והמחובר תערך על שלשה בעבור כי שלישית יוצאת משלשה והעולה הוא החשבון שאינו ידוע |
| You can also extract the two numbers. | וגם תוכל להוציא שני החשבונות |
|
אם יאמר כאשר גרענו חמישית האחד משלישית האחר נשאר ו' חברנום יעלו עשרה |
|
הדרך בזה שתחסר הנשאר מהמחובר ומן הנותר קח המחצית וככה מספר החמישית שגרע |
|
ואחר שידעת חמישיתו ידעת כלו ומן האחד תוציא השני |
| According to this way for every number. | ועל זה הדרך לכל חשבון |
|
שאם יאמר אם גרענו רביעית האחד מחמישית האחר נשאר אחד ואם חברנום יעלו י"א |
|
ככה תעשה גרע אחד מי"א ונשאר עשרה קח מחציתם והוא מספר הרביעית שגרע |
|
מתברר שהחשבון האחד הוא עשרים וממנו תוכל להוציא השני והוא שלשים |
| This way will lead you to the truth and to the correct. | וזה הדרך ידריכך אל האמת ואל הנכון |
Chapter Three: Multiplication |
השער השלישי במערכת | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| It is divided into three categories: | ונחלק לשלשה ענינים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
מערכת שלמים על שלמים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומערכת שלמים על שברים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומערכת שברים על שברים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
The First Category: [Multiplication of] Integers by Integers |
הענין הראשון בשלמים על שלמים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| You should know that every number is built and established on ten ranks: | יש לך לדעת כי כל חשבון יבנה ויכונן בעשרה מדרגות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והמדרגה הראשונה הם האחדים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והשניה העשרות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והשלישית המאות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והרביעית האלפים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והחמישית העשרות אלפים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והששית במאות אלף | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והשביעית באלף אלפים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והשמינית עשרת אלפי אלפים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והתשיעית במאת אלף אלפים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והעשירית באלף אלפי אלפים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| And so on endlessly. | וכן עד אין קץ | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Definition of the multiplication operation: the multiplication of a number by a number is that you count the first number by the multitude of the second number. | ומערכת מספר על מספר הוא שתהיה מונה המספר האחד במנין המספר השני | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Multiplication of units by units | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכל מספר שאתה עורך אותו באחד הוא עומד ואינו נוסף | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואכן אם תערוך אחד על אחד הוא אחד ושנים על אחד הם שנים וכן כל מספר שתערכנו על אחד אינו נוסף | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תערוך שנים על שנים אתה כופל אותו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם בשלשה תערכנו תמצאנו שלשה מן המספר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן עד עשרה שאם תערכנו על [22]עצמו יהיו מאה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If you multiply a number by a number that are greater than ten, you should master the multiplication of units, for every number is calculated this way. | ואם תרצה לערוך מספר על מספר למעלה מעשרה אתה צריך שיהיה חשבון האחדים סדורים על פיך כי כל חשבון מתחשב על זה הדרך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Full multiplication table: | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| To make it easier for the reader, I have drawn a table for you from 1 to 10, by which you may find out every number. | ולהקל על הקורא ציירתי לך זה המכבר מא' ועד י' שתברור לך בו כל חשבון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Instructions for using the multiplication table: | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר תרצה לערוך חשבון על חשבון מא' ועד י' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כמו שתרצה לערוך ח' על ז' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אתה נותן אצבעותיך בשני הטורים הראשונים אשר כתוב בהם מא' ועד י' על הסדר האצבע האחד על ח' ואצבעך השני על ז' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן לכל החשבון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תרצה לחשוב משאר המדרגות אחת על אחת יחשוב אותם כאלו הם אחדים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כמו שתרצה שש מאות על ארבעת אלפים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
מאיזה חשבון הם המאות מששה והאלפים מארבעה ערוך ששה על ארבעה כאלו הם אחדים והם כ"ד ושמרם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואחר כך קח מדרגת המאות והוא שלשה ומנה מהאלפים ולמעלה ג' מדרגות והוא מדרגה הששית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן אם תקח מדרגת האלפים והוא ארבעה ותמנה אתו מן המאות ולמעלה אתה מגיע אל המדרגה הששית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ושים שם חשבון האחדים ששמרת והעשרות במדרגה אשר אחריה והיא השביעית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומפני זה אתה אומר כי הכ"ד ששמרת הם ב' אלפי אלפים וארבע מאות אלף | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דרך אחרת שתהיה חושב מדרגות בב' חשבונות ותפחות אחד מהם והנשאר יהיה מדרגת המספר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כמו שתקח מדרגת המאות והוא שלשה ומדרגת האלפים ארבעה תחברם יהיו שבעה תחסר מהם אחד לעולם וישארו בידך ששה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והטעם שאתה מחסר משתי המדרגות בהתקבצם מפני שמדרגת האחד עולה לכאן ולכאן | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תערוך אחדים ועשרות על אחדים ועשרות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כמו כ"ה על כ"ח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דע מה ערך כ"ה ממאה והוא רובע קח רובע כ"ח והוא ז' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תערוך נ"ד בס"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דע מה ערך החמשים ממאה והוא חצי קח חצי מספר ששה וששים יהיו ל"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תערוך קכ"ה על עצמו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דע מה ערכו מאלף והיא שמינית קח שמינית קכ"ה והוא ט"ו וחמשה שמיניות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תערוך אחדים על עשרות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כמו חמשה על שבעים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ערוך חמשה על שבעה יעלה ל"ה יהיו העשרות מאות והאחדים עשרות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
גם תדענו שתחשוב מהו חמשה מעשרה והוא חציו קח מחצית השבעים והוא ל"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
או תדענו שתחשוב מהו השבעים ממאה והוא שבע עשיריות קח שבע עשיריות מחמשה והוא ג' וחצי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תערוך אחדים על מאות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כמו חמשה על שלש מאות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ערוך חמשה על ג' יעלה ט"ו יהיו העשרות אלפים והאחדים מאות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותדענו שתחשב מהו חמשה ממאה והוא חצי עשיריות קח חצי עשירית של ג' מאות והוא ט"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותדענו שתחלק הג' מאות על מאה ותערוך היוצא על חמשה יעלה ט"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תערוך עשרות על עשרות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כמו כ' על ל' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ערוך שנים על שלשה יהיו ששה האחדים יהיו מאות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותדענו שתחשוב מהו כ' ממאה וקח ערכו מל' ומה שיהיה תערכנו במאה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
או תדע מה שהם הל' ממאה וקח ערכו מן עשרים והדבר שוה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תערוך עשרות על מאות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כמו מ' בשש מאות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ערוך ד' על [24]ששה יעלה כ"ד יהיו האחדים אלפים והעשרות עשרות אלפים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותדענו שתחשב מהן ו' מאות מאלף והוא ו' עשיריות קח זה הערך ממ' יעלה כ"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
או תדע מהן הארבעים מאלף חלק אחד מן כ"ה וכערכו קח משש מאות והדבר שוה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תערוך עשרות על אלפים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כמו נ' בז' אלפים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וערוך ה' על זה שבעה יעלה ל"ה יהיו האחדים עשרות אלפים והעשרות מאות אלפים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותדענו שתחשב מהן ז' אלפים מי' אלפים וקח אותו הערוך מחמשים והוא ל"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
או תדע מהם הנ' מי' אלפים וכערכו קח מן הז' אלפים והדבר שוה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| By these ways you can know every number endlessly, keeping the names and the ranks. | ועל אלו הדרכים תוכל לדעת כל חשבון עד אין קץ שתשמר השמות והמדרגות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
The Second Category: Multiplication of Integers by Fractions |
הענין השני מערכת שלמים על שברים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Sexagesimal Fractions |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If you have fractions of the astrologers: degrees, minutes and seconds | אם יהיו בידך שברי חכמי המזלות מעלות ראשונים ושניים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם אתה עורך מעלות על מעל[ו]ת יהיה הנקבץ מעלות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם אתה עורך מעלות על שברים יהיה הנקבץ שברים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם ערכת על ראשונים הם ראשונים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם על שניים הם שניים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם על שלישיים הם שלישיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן למעלה מהם לכל השברים הנערכים על מעלות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כמו שתרצה לערוך כ' מעלות על נ' ראשונים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
יעלו אלף והם ראשונים תחלקם על ששים יצא בחלוק י"ו וישאר מ' ראשונים והוא ה' ששיותיו וכאותו הערך קח מן הב' יהיו י"ו מעלות מ' ראשונים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| According to this calculate the seconds, thirds and all the fractions endlessly. | ועל זה תחשב לשניים ולשלישיים ולכל השברים עד אין קץ | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Since the calculation of the integers that are multiplied by fractions is decreasing. | כי כל חשבון השלמים הנערכים בשברים הוא פחות פוחת והולך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והאומר חשוב עשרה בשליש או ברביע דומה כמי שאומר כמה חלק שליש או רביע מהעשרה וכן לכל השברים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Integers by integers and fractions |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Simple Fractions |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If you have fractions of the geometricians and you wish to multiply integers by integers and fractions. | ואם יהיו בידך שברי חכמי המדות ותרצה לערוך שלמים על שלמים ושברים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כגון שנים על שלשה ורביע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נבקש חשבון שיש לו שלישית ורביעית והוא שנים עשר והוא המורה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותקח לכל אחד שלם י"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והם כ"ד בחשבון האחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
[25]והחשבון השני השלשה יעלו ל"ו הוסף עליהם ג' בעבור הרביע יעלו ל"ט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונערוך כ"ד על ל"ט יעלו תתקל"ו חלקים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקם על קמ"ד שהוא מרובע המורה יצא ששה ונשארו ע"ב שהוא חצי קמ"ד והנה ששה וחצי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותדענו שתעזב השניים כמו שהם שנים ובעבור שבר הרביעית וקח לכל אחד מן השלשה ארבעה יעלו י"ב נוסיף אחד בעבור הרביעית הנה י"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותדענו שתקח מה שיצא ממנו הרביע והוא ארבעה והוא המורה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נערוך שנים על ד' יעלו שמונה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ושלשה על ד' יעלו י"ב ועם הרביעית י"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ערכם זה על זה יצא ק"ד וחלק על מרובע המורה שהוא י"ו יצא ששה וישאר שמונה שהוא חצי אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותדענו שתערך שנים על שלשה עלה ששה וישוב ו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וערוך על שנים על רביעית עלה שנים רביעיות שהוא חצי וזה יהיה שמור בידך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
For the one when it is multiplied by a fraction is the same [as the fraction]. 
|
כי האחד הנערך על שבר הרי הוא כמוהו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| It is like saying one in length by one-half in width, which is really nothing but one-half. The same by one-third and by all fractions. | ודומה כמי שאומר אחד באורך על חצי ברוחב וזה באמת אינו כי אם חציו וכן על שליש ועל כל השברים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Integers by fractions fractions |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If you multiply integers by fractions | ואם תערוך שלמים על שברים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Simple Fractions |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כגון שלשה רביעיות בתשעה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דע מאיזה מספר הרביעיות והוא מארבעה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלק התשעה עליהם יצא לכל אחד שנים ורובע ערוך היוצא על שלשה יהיה ששה וג' רביעיות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותדענו מאיזה מספר הרביעית והוא מארבעה והוא המורה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נערוך התשעה על ארבעה יעלה ל"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונערכם על שלשה בעבור הרביעיות שהם שלשה יעלה ק"ח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקם על י"ו שהוא מרובע המורה יצא ששה שלמים וישארו י"ב שהם ג' רביעיות אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Integers and fractions by integers and fractions |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If you multiply integers and fractions by integers and fractions | ואם תערוך שלמים ושברים על שלמים ושברים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Simple Fractions |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כגון שלשה ושביעית על חמשה ושמינית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
בקש חשבון שיש לו שני השברים כלומ' שביעית ושמינית והוא נ"ו והוא המורה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונערוך החשבון האחד שהוא שלשה על המורה ויעלה קס"ח ונוסיף עליו ח' בעבור השביעית ועלה קע"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועוד [26]נערוך החשבון האחד שהוא חמשה על המורה יעלה ר"פ ובעבור השמינית נוסיף שבעה והנה רפ"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נערוך קע"ו על רפ"ז עלה חמשים אלף וחמש מאות ושנים עשר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקנום על שלשת אלפים ומאה ושלשים ושש שהוא מרובע המורה יצא בחלוק ששה עשר ונשארו של"ו והם שלש רביעיות שביעית אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| שהאחד הוא מרובע המורה שהם ושביעיתו הם באותיות הודו ובאותיותנו הם ת'מ'ח' | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותדענו שתקח לשלשה שיש עמהם שמינית ארבעים והוסף אחד הנה מ"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ערכנום זה על זה עלו תשע מאות ושנים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקנום על נ"ו שהוא ערך שבעה על שמונה ויצא י"ו ונשארו ו' שהם שלש רביעיות שביעית אחד כי שביעית נ"ו הם ח' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותדענו שתערוך שלשה על חמשה עלה ט"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועוד תערוך ג' על שמינית עלה ג' שמיניות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וחמשה על שביעית הנה חמש שביעית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונחשוב שהם שמיניות ונחברם עם השלש עלה אחד שלם ונשאר מיתרון השביעית על השמינית חמש שמיניות שביעית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועוד יש לך לערוך שביעית על שמינית והוא שביעית שמינית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תחברם עם החמש שיש לך והנה שש שמיניות שביעית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| All of these ways yield the same. | וכל הדרכים יצאו שוה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Sexagesimal Fractions |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| For the way of the astrologers, as sixty is indivisible by seven nor by eight, do as follows: | ולדרך חכמי המזלות שלא יחלקו ששים על שבעה ולא על שמונה ככה תעשה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שתעשה מן מעלה אחת נ"ו ראשונים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומראשון נ"ו שניים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
בעבור שנ"ו יש להם שביעית ושמינית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה יש לך לערוך שלשה מעלות וח' ראשונים שהם שביעית נ"ו על חמשה מעלות ז' ראשונים שהם שמינית נ"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תערוך ג' על ה' שהם מעלות עלה ט"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותערוך השלשה שלמים על שבעה ראשונים ועלה כ"א ראשונים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואחר כן תערוך ח' על ה' שלמים יעלה מ' ראשונים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונערוך ח' ראשונים על ז' ראשונים יעלה נ"ו שניים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נחלק השניים על נ"ו עלה ראשון אשר חברנו עם הראשונים והנה ס"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נעשה מהם מעלה אחת מנ"ו עלה שש עשרה מעלות ונשארו ו' ראשונים [27]שהם ג' רביעיות משביעית נ"ו במעלה אחת | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Integers and fractions by integers and fractions of fractions |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If you multiply integers and fractions by integers and fractions of fractions | ואם תערוך שלמים ושברים על שלמים ושברי שברים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Simple Fractions |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כמו שלשה ורביעית על חמשה וששית שביעית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
קח חשבון שיש לו ששית ושביעית והוא מ"ב גם נערכנו על הארבעה בעבור הרביעית יעלו קס"ח והוא המורה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והשב כל השלמים על המורה ותחבר השברים עם כל אחד מהחשבונות והעולה תערוך זה על זה ותחלק על מרובע המורה והיוצא בחלוק הם שלמים והנשאר הם חלקים מהמורה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותדענו שתערך ג' על ה' והוא ט"ו שלמים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותערוך שלשה על ששית שביעית והוא ג' ששיות שביעית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ובעבור שתמצא כל השברים שצריכים לזה החשבון קח פ"ד שיש לו ששית ושביעית ורביעית והוא המורה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ורביעיתו כ"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ושביעיתו י"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וששיתו י"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה ג' ששיות שביעית שיצאו בערך הם ששה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ושוב וערוך רביעית על ה' והם רביעיות שהם ק"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ושוב וערוך רביעית על ששית שביעית והוא חצי אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר תחברם יעלה קי"א וחצי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
קח מהם אחד שלם שהוא פ"ד וחברהו עם הט"ו שלמים יעלו י"ו שלמים ונשארו עדין כ"ז וחצי ועשרים ואחד הוא רביעית נשאר ו' וחצי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| You can extract it in all ways. | ובכל הדרכים תוכל להוציאו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
The Third Category: Multiplication of Fractions by Fractions |
הענין השלישי מערכת שברים על שברים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Sexagesimal Fractions |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If you have fractions of the astrologers, such as minutes and seconds and you wish to multiply them by minutes and seconds. | אם יהיו בידך שברי חכמי המזלות כגון ראשונים ושניים ותרצה להעריכם על ראשונים ושניים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כשאתה עורך ראשונים על ראשונים יעלה מן הערך שניים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם ראשונים בשניים יעלה שלישיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם ראשונים בשלישים יעלה רביעים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם שלישים בשלישים אתה עורך יעלה ששיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם ברביעים יעלה שביעים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Since, every number that you multiply by fractions is decreasing from its value by the rank of the fraction by which we multiply.
|
כי כל מספר שאתה עורך אותו בשברים שברים בשברים הוא פוחת [28]ומתרחק מחשבונו כמרחק השבר אשר נערוך בו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Multiplication table of sexagesimal fractions | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| To make it easier for the reader, I have drawn a table in order to understand the product of fractions by fractions. | ולהקל על הקורא ציירתי לוח להבין העולה משברים על שברים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Instructions for using the multiplication table: when you wish to know the product of one of the types of the fractions that are written in the lines breadthwise [and lengthwise], look at where the two lines meet and this is where the product of both is. | וכאשר תרצה לדעת העולה מאחד ממיני השברים שהם כתובים בטור הרוחב הסתכל במקום אשר ייפגשו שני הטורים זה בזה ושם העולה משניהם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| When you multiply fractions by fractions: | וכאשר תערוך שברים על שברים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם יהיה העולה פחות מששים אתה מחזיק בו ומונה אותו מאותו המין היוצא מהערך ההוא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם יהיה מוסיף על ששים אתה מחלק אותו על ששים והיוצא בחלוק הוא מן המין אשר למעלה מאותו המין אשר חלקת אותו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כאלו היית עורך נ' שניים בנ' שניים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
יהיה העולה אלפים ות'ק' מאות רביעיים חלקם על ששים יצא בחלוק מ"א והם שלישים ונשארו מ' רביעיים שלא נתחלקו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| According to this way for all fractions. | ועל זה הדרך לכל השברים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Simple Fractions |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If you divide the fractions of the geometricians one by another. | ואם שברי חכמי המדות תערוך זה על זה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Fraction by Fraction |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כגון שני שלישיים על שני חמישיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| This is the sign that you keep the names of the fractions: | זה לך האות שתשמור שמות השברים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ודע כי השלישים הם חצובים משלשה והחמישיים מהחמשה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וערוך ג' על חמשה והם ט"ו והוא המורה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואחר כן תערוך שנים על שנים והם ארבעה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכערך ארבעה מט"ו כן ערך חשבון זה מאחד והוא חמישית ושליש חמישית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The reason is that saying a third by a fifth is as saying a third of a fifth, which is one part of 15.
|
והטעם כי האומר שלישי על חמישי דומה כמי שאומר שלישית מחמישית שהוא חלק אחד מט"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Or it is as saying a third lengthwise by a fifth breadthwise and it is clear that it is one part of 15. | או דומה כמי שאומר שלישי באורך על חמישי ברוחב וידוע וברור שהוא חלק אחד מט"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Fraction by fraction of fraction
|
ואם תערוך ב' חלקים מחמשה בעשירית האחד בב' שלישי אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דע כי שם החלק האחד הוא חמשים כמספר חמשה בעשרה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותערוך הנ' בג' שהוא שם החלק השני ויעלה ק"נ והוא המורה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכערך ד' שהם שני החשבונות מן ק"נ כן ערך ערכו מן האחד והוא שני שלישי [29]חמישית החמישית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Fraction of integer and fraction by fraction of integer
|
ואם תערוך שלשה רביעיות [ש]ל שלשה ושליש על ששה שביעיות חמשה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דע מאיזה מספר יצא הרביע והוא מארבעה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
קח שלשת רביעיותיו יהיו שלשה ערכם בשלשה ושליש יעלה עשרה ושמרם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואחר כך תקח ששה שביעיות חמשה והוא ארבעה וב' שביעיות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ערכם על השמור יעלה מ"ב וששה שביעיות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תחלקם על ארבעה יצא בחלוק עשרה שלמים וה' שביעיות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותדענו דע מאיזה מספר הוא השביעית והוא משבעה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
קח ששה שביעיותיו ותערכם בחמשה יעלה שלשים ושמרם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואחר כך תקח שלשה רביעיות משלשה ושליש והוא שנים וחצי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ערכם על השמור שהם ל' יעלה ע"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקם על ז' יצא בחלוק עשרה וחמשה שביעיות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותדענו שתערוך שנים וחצי שהוא החשבון האחד על ארבעה וב' שביעיות שהוא החשבון יעלה עשרה [30]וה' שביעיות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Fraction of integer and fraction by fraction of integer and fraction
|
ואם תערוך שבעה שמיניות של ה' ושליש בג' חמישיות של ו' ורובע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דע מאיזה מספר השמינית והוא משמנה קח ז' שמיניות והוא ז' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואחר כך דע מאיזה מספר החומש והוא מחמשה קח ג' חמישיותיו והם ג' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תערכם על השמור יעלה קל"ט וד' רביעיות תחלקם על שמונה יצא בחלוק שבעה עשר וחצי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
או אם תרצה תחלק השמור שהוא ל"ז ושליש על שמונה והעולה שהוא ד' וב' שלישים תערוך על י"ח ושלשה רביעיות והעולה שהוא פ"ז וחצי תחלק על חמשה יצא י"ז וחצי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותדענו שתשיב החמשה ושליש כלם שלישים יהיו י"ו שלישים קח ז' שמיניותיו והעולה שהוא י"ד ושמרם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ושוב אל הששה ורביע תשיבם כלם רביעיות ויהיו כ"ה קח שלשת חמישיותיו והעולה שהוא ט"ו תחלק על ארבעה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והיוצא בחלוק שהוא שלשה ושלשה רביעיות תערכם על השמור והעולה תחלק על שלשה יצא י"ז וחצי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
או אם תרצה תחלק השמור שהם י"ד על שלשה והיוצא שהוא ארבעה ושני שלישיים תערוך בט"ו והעולה שהוא ע' תחלק על ד' יצא י"ז וחצי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Fraction of fraction by fraction of fraction |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תערוך ג' רביעיות של ג' חומשין בה' שתותין של ג' רביעיות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דע מאיזה מספר הרובע והחומש והוא מעשרים וג' חומשיו הוא י"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואחר תקח החשבון השני ודע מאיזה מספר השתות והשביעית והוא מ"ב וכאשר תקח חמשה שתותין של שלשה שביעיות יעלה ט"ו שהם שתי שביעיות וחצי שביעית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר תערוך הב' חמישיות ורביעית חמישית השמורים על שתי שביעיות וחצי שביעית בקש לך חשבון שיהיה לו רביעית וחמישית ושביעית והוא ק"מ והוא המורה ועלה בידך ממערכת זה על זה כ"ב וחצי והם חלקים מהמורה והם שביעית ושמינית שביעית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותדענו שתדע השלשה [31]רביעיות של שלשה חומשין שהוא ט' כאשר אמרנו ואחר כך תקח חמשה שתותין של שלשה שביעיות שהם שני שביעיות וחצי שביעית ותקח מן התשעה כערכם שתי שביעיות וחצי שביעית יעלה שלשה ושביעית וחצי שביעית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תערוך שני חלקים מחמשה בעשירית האחד בשני חלקים משמונה בשלישית האחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דע שמות החלקים חלקי החשבון השני והם שמונה ושלשה ערכם זה בזה יעלה כ"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותערוך נ' בכ"ד ויעלה אלף ומאתים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכערך שמות שני החשבונות שהם שנים שנים מן אלף ומאתים כן ערכם מן האחד והוא חלק אחד משלש מאות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותדענו שתקח מן הנ' שני חלקים מחמשה בעשירית והם שנים והוא חומש החומש על רביעית השליש הוא כמי שיאמר חומש החומש מרביעית השליש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועתה בקש חשבון שליש ורביע וחומש וחומש החומש והוא שלש מאות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
קח שלישיתו והוא ק' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומן הק' רביעיתו והוא כ"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומן כ"ה חמישיתו והוא ה' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומן ה' חמישיתו והוא אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונתברר שהוא חלק אחד משלש מאות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| From these examples and ways you can understand the fractions of fractions and all of them are present for the one who understands. | ומן הדמיונות והדרכים האלה תוכל להבין בשברי השברים וכולם נכוחים למבין | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Chapter Four: Division |
השער הרביעי במחלוקת | |||||||||||||||||||||||||||||||||||||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| It is divided into three categories: | והוא נחלק לשלשה ענינים | |||||||||||||||||||||||||||||||||||||||||||||||||
[The First Category]: Division of Integers by Integers |
חלוקת שלמים על שלמים | |||||||||||||||||||||||||||||||||||||||||||||||||
Sexagesimal Fractions |
||||||||||||||||||||||||||||||||||||||||||||||||||
| If you wish to divide degrees by degrees according to the calculation of the astrologers: | אם תרצה לחלק מעלות על מעלות לחשבון חכמי המזלות | |||||||||||||||||||||||||||||||||||||||||||||||||
|
אם תחלק חשבון רב על מעט יהיה היוצא בחלוק מעלות | |||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם ישאר שלא יתחלק ערוך הנשאר על ששים יהיו ראשונים ויתחלק על מה שחלקת בראשונה והיוצא בחלוק הם ראשונים | |||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם ישאר שלא יתחלק תערכם על ששים ותחלק על מה שחלקת והיוצא הם שנים | |||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן עד כמה שתרצה | |||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם רצית לחלק חשבון מועט על רב תערוך המועט על ששים יהיו ראשונים ואחר כן [32]תחלוק והיוצא בחלוק הם ראשונים | |||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם ישאר שלא יחלק ערכם על ששים ותחלק על מה שחלקת יהיה היוצא שניים | |||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן לשלישיים ולרביעיים עד אין קץ | |||||||||||||||||||||||||||||||||||||||||||||||||
Simple Fractions |
||||||||||||||||||||||||||||||||||||||||||||||||||
| If according to the calculation of the geometricians. | ואם לחשבון חכמי המדות | |||||||||||||||||||||||||||||||||||||||||||||||||
|
תרצה לחלק חשבון רב על מועט | |||||||||||||||||||||||||||||||||||||||||||||||||
|
כגון שתרצה לחלק מאה על ט"ו | |||||||||||||||||||||||||||||||||||||||||||||||||
|
בקש חשבון שהיה מונה לשניהם והוא חמשה קח חמישית מאה והוא כ' וחלק על חמישית ט"ו והוא ג' יצא בחלוק ששה ושני שלישיות וככה מק' על ט"ו | |||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תחלק אלף על שנים ושבעים | |||||||||||||||||||||||||||||||||||||||||||||||||
|
החשבון שמונה לשניהם הוא שמונה ושמינית ע"ב תשעה ושמינית אלף קכ"ה חלק המרובה על המועט יצא בחלוק י"ד פחות תשיעית וככה מאלף על ע"ב | |||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם יהיו שני החשבונות שלא תמצא להם חשבון שמונה לשניהם | |||||||||||||||||||||||||||||||||||||||||||||||||
|
כגון שתרצה לחלק מ' על ז' | |||||||||||||||||||||||||||||||||||||||||||||||||
|
יצא בחלוק ה' וישארו לך ה' עשה מהם שביעיות יצא בחלוק ה' שלמים וה' שביעיות | |||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם מספר מועט תחלק על רב | |||||||||||||||||||||||||||||||||||||||||||||||||
|
כמו ט"ו על ק' | |||||||||||||||||||||||||||||||||||||||||||||||||
|
זה הענין יצא מהערכים | |||||||||||||||||||||||||||||||||||||||||||||||||
|
וכבר ידעת כי החמשה מונה לשניהם למספר ט"ו ג' פעם ולמספר ק' פעם וכערך ג' אל כ' שהוא עשור וחצי עשור כך ערך ט"ו אל מאה | |||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם לא תמצא מספר שיהיה מונה לשניהם | |||||||||||||||||||||||||||||||||||||||||||||||||
|
כגון ז' על מ' | |||||||||||||||||||||||||||||||||||||||||||||||||
|
קח חלק אחד מהמספר הרב ותערוך המועט אליו | |||||||||||||||||||||||||||||||||||||||||||||||||
|
ובדמיון זה תקח עשור המ' והוא ד' או חמישיתו והוא ח' או שמיניתו והוא ה' | |||||||||||||||||||||||||||||||||||||||||||||||||
|
אם עשיריתו לקחת כאשר תערוך הז' אליו תמצא בו שנים עשיריות פחות רביע העשור וכן יגיע לכל אחד מן המ' שני עשיריות אחד פחות רביע העשור | |||||||||||||||||||||||||||||||||||||||||||||||||
|
או חומש פחות שמינית החומש | |||||||||||||||||||||||||||||||||||||||||||||||||
|
או שמינית וב' חמישיות שמינית | |||||||||||||||||||||||||||||||||||||||||||||||||
|
וככה יצא בחלוק לכל אחד | |||||||||||||||||||||||||||||||||||||||||||||||||
|
ובחינתו שכשתערוך שנים עשיריות אחד פחות רביע העשור על מ' | |||||||||||||||||||||||||||||||||||||||||||||||||
|
או חומש אחד פחות שמינית החומש על מ' | |||||||||||||||||||||||||||||||||||||||||||||||||
|
או שמינית וב' חמישיות שמינית על מ' | |||||||||||||||||||||||||||||||||||||||||||||||||
|
יעלה ז' שלמים בלי [33]חסר ויתר | |||||||||||||||||||||||||||||||||||||||||||||||||
| Word Problems - Divide a Quantity Problems | ||||||||||||||||||||||||||||||||||||||||||||||||||
| Dividing a known quantity among a known number of people in given ratios | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תרצה לחלק מספר ידוע על אנשים ידועים ולתת לכל אחד ואחד כפל חברו ותרצה לדעת כמה תתן לראשון שיתחלק כל המספר בלא תוספת ובלא מגרעת | |||||||||||||||||||||||||||||||||||||||||||||||||
|
כמו שתחלק מספר ק' על ד' כל אחד ואחד כפל חבירו | |||||||||||||||||||||||||||||||||||||||||||||||||
|
זה תדע מאחד כמה יתחבר בתוספת הכפל לארבעה אנשים ועל המחובר תחלק המספר והיוצא הוא חלק הראשון | |||||||||||||||||||||||||||||||||||||||||||||||||
|
וכפל הכפל לארבעה אנשים ידוע הוא שהם ט"ו חלק עליהם הק' יצא בחלוק ששה וב' שלישיים וככה חלק הראשון | |||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן אם יאמר להוסיף לשני חצי הראשון ולשלישי שלישית השני ולרביעית רביעית השלישי | |||||||||||||||||||||||||||||||||||||||||||||||||
|
תדע זה מאחד כמה יתחבר עד ארבעה וידוע הוא שיתחבר ז' שלמים | |||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||
|
תחלק עליהם הק' יצא בחלוק י"ד ושתי שביעיות וככה חלק הראשון | |||||||||||||||||||||||||||||||||||||||||||||||||
| According to this way for every number whether great or small. | ועל זה הדרך לכל מספר בין רב למעט | |||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תחלק ק' על ב' והיוצא בחלוק האחד תחלק על ג' והיוצא על ד' והיוצא על ה' ותרצה לדעת כמה חלק החמישי | |||||||||||||||||||||||||||||||||||||||||||||||||
|
ערוך השנים בשלשה והעולה בד' והעולה בה' יעלה ק"כ | |||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר תחלק הק' על העולה שהוא ק"כ יצא אחד פחות שתות וככה חלק החמישי | |||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תחלק צ' על ט' אנשים ושיוסיף כל אחד על חברו אחד כמה יתן לראשון ויצא שוה | |||||||||||||||||||||||||||||||||||||||||||||||||
|
זה הכלל יהיה בידך שתגרע אחד מחשבון האנשים והנשאר שהם שמונה דע כמה יתחבר מאחד ועד שמונה והוא שתוסיף אחד על שמונה והמחובר תערוך על חצי השמונה יעלה ל"ו | |||||||||||||||||||||||||||||||||||||||||||||||||
|
גרע אותם מהתשעים והנשאר שהוא ארבעה וחמשים חלקם על התשעה והיוצא שהוא שוה ששה הוא חלק הראשון | |||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה הכלל ידריכך בכל השאלות הדומות לזו שיהיו כל התוספות שוות שיהיה תוספת השלישי על השני כתוספת רביעי על השלישי והחמישי על הרביעי וכן כלם | |||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תחלק שמונים על חמשה אנשים ושיוסיף השני על הראשון ג' והשלישי על השני אחד והרביעי על השלישי שנים והחמישי על הרביעי ששה ותרצה לדעת כמה יתן לראשון | |||||||||||||||||||||||||||||||||||||||||||||||||
|
חבר [34]כל התוספות שיש על הראשון יעלו כ"ה וגרעם מהשמונים והנשאר שהוא נ"ה חלק על האנשים יצא בחלוק י"א וככה חלק הראשון | |||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תחלק מספר ידוע לשלשה אישים ותרצה לדעת כמה נתן לכל אחד | |||||||||||||||||||||||||||||||||||||||||||||||||
|
ככה תעשה שתצוה לו לכפול חלק הראשון וחלק השני כלו יערוך על המספר פחות אחד וחלק השלישי על המספר כלו ויחבר הכל | |||||||||||||||||||||||||||||||||||||||||||||||||
|
ואחר כן תשאל ממנו כמה יש להשלמת מרובע החשבון אשר חילק | |||||||||||||||||||||||||||||||||||||||||||||||||
|
ומה שיהיה חלק על המספר ההוא אשר חילק פחות שנים והיוצא בחלוק הוא חלק הראשון | |||||||||||||||||||||||||||||||||||||||||||||||||
|
ומה שישאר ולא יוכל לחלקו עד שיעשנו ש[למ]ים הוא חלק השני ו[מז]ה תדע השלישי | |||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה החשבון פעמים שישתבש שיצא הכל בחלוק | |||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר יקרך זה תדע שלא נתן לראשון כי אם אחד בלבד והנשאר כלו תן לשני ומהם תדע השלישי | |||||||||||||||||||||||||||||||||||||||||||||||||
The Second Category: Division of Fractions by Integers or Integers by Fractions |
הענין השני חלוקת שברים על שלמים או שלמים על שברים | |||||||||||||||||||||||||||||||||||||||||||||||||
Sexagesimal Fractions |
||||||||||||||||||||||||||||||||||||||||||||||||||
| If you have sexagesimal fractions [lit. fractions of the astrologers] and you wish to divide them by degrees, the result of division will be of the same type of the fractions: if they are primes, [the result will be] primes; if seconds [the result are] seconds; and so on for all the fractions | אם יהיו בידך שברי חכמי המזלות ותרצה לחלקם על מעלות יהיה היוצא בחלוק ממין השבר אם ראשונים ראשונים ואם [שניים] שניים וכן לכל השברים | |||||||||||||||||||||||||||||||||||||||||||||||||
| Because, for every fraction you multiply by a degree, the result is of the same type of that fraction. | מפני שכל שבר אשר אתה עורך אותו במעלה יהיה העולה ממין השבר ההוא | |||||||||||||||||||||||||||||||||||||||||||||||||
| If something remains that cannot be divided, multiply the remainder by sixty and divide by what you have divided; the result will be fractions that are lower than the first type by one rank.
|
ואם ישאר שלא יתחלק תערוך הנשאר על ששים ותחלק על מה שחלקת והיוצא יהיו שברים יורדים מדרגה אחת מן המין הראשון | |||||||||||||||||||||||||||||||||||||||||||||||||
| If you divide degrees by [sexagesimal] fractions, you convert the degrees into the type by which you want to divide, then divide this type by its own type and the result of division is degrees.
|
ואם תחלק מעלות על שברים אתה משיב המעלות אל המין אשר אתה רוצה לחלוק עליו ותחלק מין על מינו ויהיה היוצא בחלוק מעלות | |||||||||||||||||||||||||||||||||||||||||||||||||
| You must always convert the degrees into the type by which you want to divide, even if the degrees are many. | ואתה צריך לעולם להשיב המעלות אל המין אשר תרצה לחלק עליו וגם אם יהיו המעלות רבות | |||||||||||||||||||||||||||||||||||||||||||||||||
|
כגון שתרצה לחלק עשרה מעלות על חמשה ראשונים | |||||||||||||||||||||||||||||||||||||||||||||||||
|
אי איפשר לומ' שתחלק עשרה על חמשה ויצא בחלוק ב' מעלות | |||||||||||||||||||||||||||||||||||||||||||||||||
|
כי כאשר תערוך מעלות על ראשונים יהיו ראשונים כאשר אמרנו בשער המגרעת | |||||||||||||||||||||||||||||||||||||||||||||||||
|
[35]וכל חשבון כאשר תערוך מה שיצא בחלוק על הנחלק עליו יעלה החשבון המחולק | |||||||||||||||||||||||||||||||||||||||||||||||||
|
ומפני זה אתה עורך העשרה על ששים יעלו ת"ר ותחלק על חמשה יצא בחלוק ק"כ והם מעלות | |||||||||||||||||||||||||||||||||||||||||||||||||
|
כי המחלק עשרה מעלות על ה' ראשונים כך הוא רוצה לעשות שיחלק הי' מעלות שלא יהיה בצד האחד שהוא הרחב כי אם ה' ראשונים ותמצא שיהיה באורך ק"כ מעלות | |||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם ישאר שלא יתחלק תערוך הנשאר על ששים ותחלק מה שחלקת והיוצא יהיו שברים יורדים מדרגה מן המין הראשון | |||||||||||||||||||||||||||||||||||||||||||||||||
Simple Fractions |
||||||||||||||||||||||||||||||||||||||||||||||||||
| If you divide simple fractions [lit. fractions of the geometricians]: | ואם שברי חכמי המדות תחלק | |||||||||||||||||||||||||||||||||||||||||||||||||
Integer by integer and fraction |
||||||||||||||||||||||||||||||||||||||||||||||||||
|
כגון מי שמחלק ק' על שנים ורביע | |||||||||||||||||||||||||||||||||||||||||||||||||
|
דע מה ערך האחד מן השנים ורביע והוא ארבע תשיעיות | |||||||||||||||||||||||||||||||||||||||||||||||||
|
וקח ארבעת תשיעיות הק' שהם מ"ד שלמים וד' תשיעיות וכן יגיע לכל אחד מהשנים ורביע | |||||||||||||||||||||||||||||||||||||||||||||||||
Integer by fraction of integer and fraction |
||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תחלק עשרים על שני שלישי שבעה וחומש | |||||||||||||||||||||||||||||||||||||||||||||||||
|
דע כמה שני שלישי ז' וחומש והוא ארבעה וארבע חומשין שהם כ"ד חומשין | |||||||||||||||||||||||||||||||||||||||||||||||||
|
ודע מה ערך האחד מהם והוא שתות ורובע השתות | |||||||||||||||||||||||||||||||||||||||||||||||||
|
וקח מהעשרים שתותם ורובע שתותם והם ארבעה ושתות וככה חלק כל אחד | |||||||||||||||||||||||||||||||||||||||||||||||||
Integer and fraction by fraction |
||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תחלק ב' על ג' שביעיות | |||||||||||||||||||||||||||||||||||||||||||||||||
|
דע בכמה ישלמו הג' שביעיות עד שי' שיהיו אחד והוא שנים פעמים ושליש פעם | |||||||||||||||||||||||||||||||||||||||||||||||||
|
ערכם בעשרים שביעיות יעלה ז' פחות שליש וככה חלק כל אחד | |||||||||||||||||||||||||||||||||||||||||||||||||
Integer and fraction by fraction and fraction of fraction |
||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תחלק עשרים על ז' שמיניות וחצי שמיניות | |||||||||||||||||||||||||||||||||||||||||||||||||
|
דע בכמה ישלמו הז' שמיניות וחצי שמיניות עד שיהיו אחד והוא אחד ושליש חומש | |||||||||||||||||||||||||||||||||||||||||||||||||
|
תערכם על עשרים שמיניות יעלה כ"א שמיניות ושליש שהם שנים וה' שמיניות ושלש שמינית שהם ג' פחות שליש | |||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||
Completion of Fractions |
||||||||||||||||||||||||||||||||||||||||||||||||||
| The way to know the multiplicative inverses of fractions so that they become one: | ודרך לדעת תשלומי השברים עד אשר יהיו אחד ככה תדענו | |||||||||||||||||||||||||||||||||||||||||||||||||
|
כגון שתרצה לדעת איך ג' שמיני האחד ישלמו עד שיהיו אחד שלם | |||||||||||||||||||||||||||||||||||||||||||||||||
|
דע כי שם השמיניות הוא משמונה ומספר הפעמים אשר השלשה מונים את השמונה הם ג' פעמים פחות שליש [36]וכמספר הזה אתה חושב את שלשת השמיניות ויהיו אחד | |||||||||||||||||||||||||||||||||||||||||||||||||
| For The number of times that one number [i.e., the numerator] counts the denominator is the same as [the number of times that] the part counts the one.
|
כי כמנין הפעמים אשר מספר אחד מונה את המספר המוכיח כמוהם החלק מונה את האחד | |||||||||||||||||||||||||||||||||||||||||||||||||
|
כגון שתרצה להשלים ב' חמישיות ועשירית החומש עד שיהיו אחד | |||||||||||||||||||||||||||||||||||||||||||||||||
|
בקש חשבון שיהיה לו חמישית ועשירית והוא נ' והוא המוכיח אשר נקרא בספר הזה המורה | |||||||||||||||||||||||||||||||||||||||||||||||||
|
וב' חמישי המספר הזה ועשירית חמישיתו הוא כ"א | |||||||||||||||||||||||||||||||||||||||||||||||||
|
והמספר הזה מונה את החמשים ב' פעמים ושליש פעם ושביעית השליש וכענין הזה יהיו תשלומי ב' חמישיות ועשירית החומש ויהיה אחד | |||||||||||||||||||||||||||||||||||||||||||||||||
| For an integer you can also say the same way. | וגם במספר השלם אתה יכול לומר על זה הדרך | |||||||||||||||||||||||||||||||||||||||||||||||||
|
כגון שתרצה להשלים את השבעה עד שיהיו י"ו | |||||||||||||||||||||||||||||||||||||||||||||||||
|
אתה יודע כי השבעה מונה את י"ו שני פעמים ושני שביעי פעם ובהם ישלמו י"ו | |||||||||||||||||||||||||||||||||||||||||||||||||
|
ועל זה הדרך תוכל למצא לכל חשבון | |||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תחלק שלשה רביעיות של שלשה חומשי תשעה על כ"ד | |||||||||||||||||||||||||||||||||||||||||||||||||
|
וידוע כי שלשה חומשי תשעה הם כ"ז חומשין ושלשה רביעיותיהם הם ד' שלמים וחצי עשירית אחד | |||||||||||||||||||||||||||||||||||||||||||||||||
|
ולפיכך אמ' המחבר תקח וכו' | |||||||||||||||||||||||||||||||||||||||||||||||||
|
תקח חלק אחד מכ"ד שמיניתו והוא ג' או שלישיתו והוא ח' או רביעיתו והוא ו' | |||||||||||||||||||||||||||||||||||||||||||||||||
|
אם שמיניתו לקחת כאשר תערוך הד' וחצי עשירית אחד אליו תמצא בו פעם אחת שמינית ושליש שמינית וחצי עשירית שליש שמינית | |||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם שלישיתו לקחת תמצא בו חצי שלישיתו וחצי עשירית שמינית שלישית | |||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם רביעיתו לקחת תמצא בו שני שלישי שלישית וחצי עשירית ששית רביעית | |||||||||||||||||||||||||||||||||||||||||||||||||
|
וככה יצא בחלוק לכל אחד מכ"ד | |||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||
|
והבחינה כשתערוך איזה שתרצה מהחלקים שהזכרנו על כ"ד יעלה ד' וחצי עשירית אחד | |||||||||||||||||||||||||||||||||||||||||||||||||
The Third Category: Division of Fractions by Fractions |
הענין השלישי חלוקת שברים על שברים | |||||||||||||||||||||||||||||||||||||||||||||||||
Sexagesimal Fractions |
||||||||||||||||||||||||||||||||||||||||||||||||||
| The first rule | ||||||||||||||||||||||||||||||||||||||||||||||||||
| If you divide sexagesimal fractions [lit. fractions of the astrologers] by each other: | אם שברי חכמי המזלות תחלק זה על זה | |||||||||||||||||||||||||||||||||||||||||||||||||
|
אם ראשונים על ראשונים תחלק יהיה היוצא בחלוק מעלות | |||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן אם שניים על שניים תחלק היוצא מעלות | |||||||||||||||||||||||||||||||||||||||||||||||||
| The same for the rest of the [sexagesimal] fractions: if you divide them by their type, the result will be degrees - this is the first rule of the division of [sexagesimal] fractions.
|
וכן שאר [37]השברים אם אתה מחלק אותם על מיניהם יהיה היוצא מעלות וזה כלל אחד בחלוק השברים | |||||||||||||||||||||||||||||||||||||||||||||||||
| The second rule | ||||||||||||||||||||||||||||||||||||||||||||||||||
| The second rule: know that if you divide one type of fractions by another type that is lower: | וכלל שני דע שאם אתה מחלק מין אחד מן השברים על מין אחר שהוא תחתיו | |||||||||||||||||||||||||||||||||||||||||||||||||
|
כגון שתרצה לחלק ראשונים על שניים או על שלישיים | |||||||||||||||||||||||||||||||||||||||||||||||||
|
אתה עורך הראשונים על ששים ויהיו שניים ותחלק שניים על שניים | |||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם על שלישיים תחלק ערכם על ששים פעם אחרת ויהיו שלישיים ותחלק שלישיים על שלישיים ויהיה היוצא מעלות | |||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן כל הדומה לזה | |||||||||||||||||||||||||||||||||||||||||||||||||
| The third rule | ||||||||||||||||||||||||||||||||||||||||||||||||||
| The third rule: if you divide one type of fractions by another type higher than it, the result of division will be of a lower type than the type [that is divided] according to the type by which you divide. | וכלל שלישי אם תחלק מין אחד מן השברים על מין אחר שהוא גדול ממנו יהיה היוצא בחלוק מן המין אשר תחתיו של המין אשר אתה מחלק עליו | |||||||||||||||||||||||||||||||||||||||||||||||||
|
כאלו חלקת שלישיים על ראשונים יהיה היוצא בחלוק שניים | |||||||||||||||||||||||||||||||||||||||||||||||||
|
או שניים על ראשונים יהיו ראשונים | |||||||||||||||||||||||||||||||||||||||||||||||||
| Because, when you multiply the result of division by the divisor, the result should be the original dividend.
|
מפני שכשתערוך מה שיצא בחלוק על מה שחלקת עליו צריך שיצא הראשון הנחלק | |||||||||||||||||||||||||||||||||||||||||||||||||
| Remainder | ||||||||||||||||||||||||||||||||||||||||||||||||||
| If something remains that cannot be divided, you multiply the remainder by sixty, then divide by what you have divided; the result will be of a type that is one rank lower than the type of the number that initially resulted from the division. | ואם ישאר שלא יתחלק אתה עורך הנשארים בששים ותחלק על מה שחלקת ויהיה היוצא ממין שירד מדרגה אחת ממין המספר שיצא בחלוק בראשונה | |||||||||||||||||||||||||||||||||||||||||||||||||
|
כאלו תחלק חמשים ראשונים על שבעה ראשונים | |||||||||||||||||||||||||||||||||||||||||||||||||
|
יצא בחלוק שבע מעלות וישאר שלא יתחלק אחד | |||||||||||||||||||||||||||||||||||||||||||||||||
|
ערכהו על ששים יהיו שניים | |||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקם על שבעה אשר חלקת בראשונה יצא בחלוק שמונה ראשונים וישאר שלא יתחלק ד' שניים | |||||||||||||||||||||||||||||||||||||||||||||||||
|
ערכם על ששים יעלו ר"מ שלישיים | |||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקם על ז' יעלה ל"ד שניים וישאר שני שלישיים | |||||||||||||||||||||||||||||||||||||||||||||||||
|
ערכם בששים וחלקם על מה שחלקת ויעלו שלישיים | |||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן אתה יכול לחקרן לרביעיים ולחמישיים עד כמה שתרצה | |||||||||||||||||||||||||||||||||||||||||||||||||
| division table of sexagesimal fractions | ||||||||||||||||||||||||||||||||||||||||||||||||||
| To make it easier for the reader, I have drawn for you a table of primes to sixths. | ולהקל על הקורא ציירתי לך לוח מראשונים עד ששיים | |||||||||||||||||||||||||||||||||||||||||||||||||
| ||||||||||||||||||||||||||||||||||||||||||||||||||
| ||||||||||||||||||||||||||||||||||||||||||||||||||
| If you want to divide degrees and fractions by degrees and fractions: convert the two numbers into one rank, then divide. | ואם תרצה לחלק מעלות ושברים על מעלות ושברים השב שני החשבונים למדרגה אחת ואחר תחלק | |||||||||||||||||||||||||||||||||||||||||||||||||
|
כגון שתרצה לחלק שמנה מעלות ועשרים ראשונים על ג' [38]מעלות וט"ו ראשונים | |||||||||||||||||||||||||||||||||||||||||||||||||
|
נשיבם כלם ראשונים והנה החשבון האחד ת"ק והוא המחולק והשני קצ"ה חלקנו הגדול על הקטן יצא בחלוק שנים והם מעלות ונשארו ק"י | |||||||||||||||||||||||||||||||||||||||||||||||||
|
ערוך אותם על ששים וחלק על קצ"ה יצא בחלוק ל"ג והם ראשונים וישאר קס"ה | |||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||
|
ערכם על ס' וחלק על קצ"ה ראשונים יצא בחלוק נ' והם שניים | |||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||
| If you want to make it more accurate with thirds and fourths, do it in this way. | ואם תרצה לדקדק כן לשלישיים ולרביעיים תעשה על זה הדרך | |||||||||||||||||||||||||||||||||||||||||||||||||
|
או תדע מה ערך ק"י הנשארים אל ש"צ הנחלקים והנה רביעיתם צ"ז וחצי נשארו י"ב וחצי והו' וחצי הם שלישית חמישית הרביעית ונשארו ו' שהם ו' חלקים מי"ג בשני שלישי חמישית רביעית | |||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||
|
ובעבור הרביעית הוסף רביעית ב' מעלות שיצאו בחלוק והם ל' ראשונים ובעבור ו' חלקם מי"ג בשני שלישי חמישית רביעית הוסף ראשון אחד לשניים והנה יצא בחלוק ל"ג ראשונים נ' שניים כמו החשבון הראשון | |||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||
Simple Fractions |
||||||||||||||||||||||||||||||||||||||||||||||||||
Integer and fraction by integer and fraction |
||||||||||||||||||||||||||||||||||||||||||||||||||
| If you divide simple fractions [lit. fractions of the geometricians]: | ואם שברי חכמי המדות תחלק | |||||||||||||||||||||||||||||||||||||||||||||||||
|
כגון שלשה ושלשה רביעיות על אחד וחומש | |||||||||||||||||||||||||||||||||||||||||||||||||
|
דע מה ערך האחד מן אחד וחומש והוא חמשה שתותיו | |||||||||||||||||||||||||||||||||||||||||||||||||
|
קח ה' שתותין מג' וג' רביעיות אחד שהם שש שמיניות יעלה [39]שלשה ושמינית וככה חלק האחד | |||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||
Integer and fraction by fraction |
||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תחלק ז' וחומש על שלש רביעיות אחד | |||||||||||||||||||||||||||||||||||||||||||||||||
|
דע בכמה ישלמו הג' רביעיות לאחד כלו' שתוסיף על ז' וחומש שלישיתם והוא אחד ושליש | |||||||||||||||||||||||||||||||||||||||||||||||||
|
תערכם על ז' וחומש יעלה תשעה וג' חומשין ורביעיתם הוא חלק כל רביע ורביע | |||||||||||||||||||||||||||||||||||||||||||||||||
|
או תשיב הז' וחומש לחומשין יהיו בידך ל"ו חומשין | |||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקם על הג' רביעיות יצא בחלוק י"ב חומשין שהם שנים וב' חומשין לכל רביע ורביע שהוא לאחד שלם ט' וג' חומשין | |||||||||||||||||||||||||||||||||||||||||||||||||
Fraction by fraction |
||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תחלק ששה שביעיות על שמונה עשיריות | |||||||||||||||||||||||||||||||||||||||||||||||||
|
דע בכמה ישלים אחד והוא אחד ורביע | |||||||||||||||||||||||||||||||||||||||||||||||||
|
תערכם על ששה שביעיות יעלה א' שביעיות וחצי | |||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקם יצא אחד וחצי שביעית וככה חלק האחד | |||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||
|
או אם תרצה חלק ששה על שמונה יצא אחד פחות רובע | |||||||||||||||||||||||||||||||||||||||||||||||||
|
לפיכך יצא עכשו שביעית אחד פחות רובע שביעית לחלק העשירית והם י' לאחד ז' שביעיות וחצי שביעית | |||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||
Fraction of integer and fraction by fraction of integer and fraction |
||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תחלק ששה שביעיות חמשה ורובע על שלשה שמיניות מאחד וחומש | |||||||||||||||||||||||||||||||||||||||||||||||||
|
השב החמשה ורובע כלם לרביעיות יעלו כ"א וששה שביעיותיו י"ח שהם ד' שלמים וחצי | |||||||||||||||||||||||||||||||||||||||||||||||||
|
ועוד השב האחד וחומש לחמישיות והם ששה חמישיות ושלשה שמיניותיו ב' חומשין ורביע חומש | |||||||||||||||||||||||||||||||||||||||||||||||||
|
ותדע בכמה יהיו אחד והוא בשנים ושני תשיעיותיו | |||||||||||||||||||||||||||||||||||||||||||||||||
|
כי רביעית חומש הוא תשיעית ב' חומשין ורביע חומש | |||||||||||||||||||||||||||||||||||||||||||||||||
|
תערכם בארבעה וחצי כלו' תקח שני פעמים ד' וחצי ותקח שני תשיעיותיהם יעלה עשרה והם ה' חומשין נמצא מגיע שנים שלמים לכל חומש וחומש ומהם תדע חלק אחד שלם | |||||||||||||||||||||||||||||||||||||||||||||||||
Chapter Five: Ratios |
השער החמישי בערך | ||||||||||||||||||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
Sexagesimal Fractions |
|||||||||||||||||||||||||||||||
| The method of the astrologers is to calculate all their calculations by sixty, since it has most of the divisors. | דרך חכמי המזלות לחשוב כל חשבונם על ששים מפני שיש לו רוב החלקים | ||||||||||||||||||||||||||||||
|
רצינו לערוך שלשים על עשרים ולחלק על ששים | ||||||||||||||||||||||||||||||
|
דע מה ערך החשבון האחד שהוא שלשים | ||||||||||||||||||||||||||||||
|
או דע מה ערך עשרים אל ששים והוא שלישיתו תקח שלישית החשבון האחר והנה עשרה | ||||||||||||||||||||||||||||||
|
ואם החשבון האחד נ' והשני מ' ונחלק העולה על ששים | ||||||||||||||||||||||||||||||
|
קח ערך נ' אל ששים והוא חמש ששיות וכזה הערך מארבעים והנה מששה ושלשים קח חמש ששיותיו והם שלשים ונשארו ארבעה | ||||||||||||||||||||||||||||||
|
ערכם על חמשה עלו עשרים | ||||||||||||||||||||||||||||||
|
תחלקם על ששה יצא שלשה שלמים ושליש שהם עשרים שברים והנה המספר ל"ג ושליש | ||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||
|
או קח ערך מ' אל ס' והוא שני שלישיותיו | ||||||||||||||||||||||||||||||
|
קח שני שלישיות נ' והנה מן מ"ח שנים ושלשה ונשארו שנים | ||||||||||||||||||||||||||||||
|
נערכים על שנים שהם שתי שלישיות ועלו ארבעה | ||||||||||||||||||||||||||||||
|
נחלקם על שלשה יצא אחד ושליש | ||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||
|
ואם שני חשבונים שאין לאחד מהם ערך אל ששים כמו י"ד ול"ט | ||||||||||||||||||||||||||||||
|
ככה נעשה נוסיף על חשבון י"ד חלק אחד והנה ערכו רביעית | ||||||||||||||||||||||||||||||
|
וככה נוסיף על ל"ט אחד ויהיה רביעיתו עשרה | ||||||||||||||||||||||||||||||
|
ויש לנו לחסר שני חסרונים כי שנים הוספנו והנה בעבור האחד שהוספנו על ל"ט יש לנו לחסר חמשה עשר שניים ונערוך האחד שהוספנו על ל"ט ויעלו ל"ט שניים | ||||||||||||||||||||||||||||||
|
נחברם עם ט"ו שהיו לנו עלו נ"ד | ||||||||||||||||||||||||||||||
|
נחסרם מראשון אחד ועלה החשבון ט' ראשונים גם ו' שניים | ||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||
|
או נוסיף על ל"ט אחד | ||||||||||||||||||||||||||||||
|
ודע מה ערכו אל ששים והנו שתי שלישיות | ||||||||||||||||||||||||||||||
|
והנה נקח שתי שלישיות ארבעה עשר והנו ט"כ | ||||||||||||||||||||||||||||||
|
כי מט"ו יהיו שתי שלישיות עשרה | ||||||||||||||||||||||||||||||
|
ויש לנו לחסר שתי שלישיות ראשון אחד שהם מ' שניים והנה המספר ט"כ | ||||||||||||||||||||||||||||||
|
ובעבור שהוספנו על ל"ט אחד נערכנו על י"ד ויעלו י"ד שניים | ||||||||||||||||||||||||||||||
|
נחסרם מעשרים נשארו לך ו' שניים | ||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||
|
ואם רצינו לערוך ל"ה על שלשה עשר ונחלק על ששים | ||||||||||||||||||||||||||||||
|
בקשנו מה ערך שנים עשר שהוא הקרוב אל י"ג אל ששים והוא חמישיתו | ||||||||||||||||||||||||||||||
|
לקחנו חמישית ל"ה והנה שבעה | ||||||||||||||||||||||||||||||
|
ונשאר אחד ערכנוהו על ל"ה והנם שניים | ||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||
|
והבחינה ידענו כי ל"ה שלש חמישיות בתוספת [41]אחד | ||||||||||||||||||||||||||||||
|
לקחנו ג' חמישיות חמשה עשר שהוא המספר הקרוב אל י"ג והיו ט' | ||||||||||||||||||||||||||||||
|
והוספנו שנים כי י"ג היו לנו ערכנום על ל"ה והוא חלק אחד ועשרה שניים | ||||||||||||||||||||||||||||||
|
והוספנו בתחלה אחד כי לקחנו ששה ושלשים והיו ט"ו | ||||||||||||||||||||||||||||||
|
חסרנו הכל מתשעה ונשאר החשבון | ||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||
|
או לקחנו חצי י"ג בעבור השלשים והנם ששה וחצי שהוא שלשים שניים | ||||||||||||||||||||||||||||||
|
וערכנו חמשה על י"ג עלה חלק אחד וחמשה שניים | ||||||||||||||||||||||||||||||
|
חברנום ועלה המספר | ||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||
|
ואם שני חשבונים האחד מ"ו והשני כ"ה | ||||||||||||||||||||||||||||||
|
והנה לא מצאנו ערך למ"ו עם ששים | ||||||||||||||||||||||||||||||
|
נחסר אחד מהחשבון ישאר מ"ה וערכו שלש רביעיות | ||||||||||||||||||||||||||||||
|
ונקח מכ"ה כ"ד ושלש רביעיותיו י"ח נשארו ג' רביעיות אחד שהם מ"ה שניים | ||||||||||||||||||||||||||||||
|
וערכנו האחד שיש תוספת על מ"ה על כ"ה ועלה כ"ה | ||||||||||||||||||||||||||||||
|
הוספנום על מ"ה שניים שהיו לנו ועלה ראשון אחד ונשארו עשרה שניים והנה החשבון י"ט י' | ||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||
|
או נקח מה ערך כ"ה אל ס' והיו שלישיתו ורביע שלישיתו | ||||||||||||||||||||||||||||||
|
נקח ממ"ו שלישיתו שהוא ט"ו ועשרים שניים | ||||||||||||||||||||||||||||||
|
ורביעית ט"ו ועשרים שניים הם שלשה וגם נ' שברים | ||||||||||||||||||||||||||||||
|
חברנום עם אשר למעלה עלה י"ט י' | ||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||
|
או נחסר אחד מכ"ה ונדע מה ערך כ"ד אל ששים והנו שתי חמישיות | ||||||||||||||||||||||||||||||
|
ושתי חמישיות מ"ה הם י"ח | ||||||||||||||||||||||||||||||
|
והאחד שנשאר להשלמת כ"ה נערכנו על מ"ה יהיו שניים | ||||||||||||||||||||||||||||||
|
גם נערוך האחד שהוא תוספת על כ"ה | ||||||||||||||||||||||||||||||
|
ונחבר כל השניים ועלה המספר | ||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||
|
או נוסיף על מ"ו שנים ועלה מ"ח וערכו אל ששים ארבע חמישיות | ||||||||||||||||||||||||||||||
|
נקח ארבע חמישיות כ"ה והנם עשרים | ||||||||||||||||||||||||||||||
|
ואנחנו הוספנו שנים נערכם על כ"ה עלו נ' שניים | ||||||||||||||||||||||||||||||
|
נחסרם מעשרים ישאר י"ט | ||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||
| Always do as follows: find a number that has a ratio to sixty, or an approximate ratio, whether by adding to it, or by subtracting. Take the ratio from that number. Subtract finally if you added at first, or add finally if you subtracted at first.
|
וככה תעשה לעולם שהסתכל איזה חשבון יש לו ערך אל ששים או ערך קרוב אליו בין להוסיף עליו בין לגרוע ומאותו החשבון תקח הערך ואם הוספת בראשונה גרע באחרונה ואם גרעת בראשונה תוסיף [42]באחרונה | ||||||||||||||||||||||||||||||
| If one of the numbers is greater than the divisor, or both are greater than it, find the ratio of the divisor to one of the numbers and take its ratio from the other number.
|
ואם היה אחד החשבונים גדול מהמחולק עליו או שניהם גדולים ממנו הסתכל מה ערך חשבון שיחלק עליו אל אחד החשבונים וכערכו קח מן החשבון האחר | ||||||||||||||||||||||||||||||
|
כגון שמונים על תשעים מחולקים על ששים | ||||||||||||||||||||||||||||||
|
נסתכל מה ערך ששים אל שמונים והוא כמהו ושלישיתו ונוסיף על תשעים שלישיתו יעלו מאה ועשרים | ||||||||||||||||||||||||||||||
|
או הסתכל מה ערך ס' אל צ' והוא כמוהו וחציו והנה נוסיף על פ' חציו יעלו ק"כ | ||||||||||||||||||||||||||||||
|
ואם החשבון האחד פ' והשני פ"ח | ||||||||||||||||||||||||||||||
|
נחשוב שהוא צ' מפני שיש לו ערך אל ס' ויצא החשבון ק"כ | ||||||||||||||||||||||||||||||
|
ובעבור שהוספנו שנים נערכם על שמונים שהוא החשבון האחר יעלו מאה וששים ראשונים והם שתי מעלות מ' ראשונים | ||||||||||||||||||||||||||||||
|
חסרם מק"כ ישאר קי"ז כ' וככה החשבון | ||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||
Simple Fractions |
|||||||||||||||||||||||||||||||
| The geometricians said that there are five kinds of ratios and they are derived from the units and they are: | וחכמי המדות אמרו שחמשה ערכים הם ונלקחים מן האחדים והם | ||||||||||||||||||||||||||||||
| The first is the multiple ratio:
|
האחד ערך הכפל | ||||||||||||||||||||||||||||||
|
כמו שנים או שלשה עם אחד | ||||||||||||||||||||||||||||||
| The second is the superparticular ratio [lit. the same and a part]:
|
והשני כמוהו וחלק ממנו | ||||||||||||||||||||||||||||||
|
כמו שנים עם שלשה וזה הערך לא ימצא קודם זה | ||||||||||||||||||||||||||||||
| The third is the multiple superparticular ratio:
|
והשלישי כפלו וחלק ממנו | ||||||||||||||||||||||||||||||
|
כמו חמשה עם שנים גם הוא | ||||||||||||||||||||||||||||||
| The fourth ratio is the superpartient ratio [lit. the same and parts]:
|
הערך הרביעי שהוא כמוהו וחלקים ממנו | ||||||||||||||||||||||||||||||
|
והיו כמו חמשה עם שלשה | ||||||||||||||||||||||||||||||
| The fifth is the multiple superpartient ratio:
|
והחמישי שהוא הכפל וחלקים ממנו | ||||||||||||||||||||||||||||||
|
לא ימצא עד שמונה עם שלשה | ||||||||||||||||||||||||||||||
| There are no ratios other than these. | ואין ערכים אלא אלו | ||||||||||||||||||||||||||||||
| All kinds of ratios are divided into three categories of progressions: | |||||||||||||||||||||||||||||||
| The science of ratio is according to three ways: | וחכמת הערכין על שלש דרכים | ||||||||||||||||||||||||||||||
| Arithmetic Progression:
|
|||||||||||||||||||||||||||||||
|
האחת כמו ארבעה וששה ושמונה שהתוספת היא שוה והוא דרך החשבון | ||||||||||||||||||||||||||||||
| Geometric Progression
|
|||||||||||||||||||||||||||||||
|
והשנית כמו ד' ו' ט' שערך ו' אל ד' כמהו וחציו וככה ערך ט' אל ו' | ||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||
|
או תוכל לומ' כי ערך ד' אל ו' שתי שלישיותיו וככה ו' עם ט' | ||||||||||||||||||||||||||||||
|
ולעולם אם ערכנו קטן על גדול יהיה כמרובע התיכון | ||||||||||||||||||||||||||||||
|
וככה אם לקחנו ארבעה ומספרים שיהיה ערך הרביעי אל השלישי כערך השני אל הראשון | ||||||||||||||||||||||||||||||
|
אם ערכנו הראשון על הרביעי יהיה המחובר כמחובר העולה מערך השני על השלישי | ||||||||||||||||||||||||||||||
| Harmonic Progression
|
|||||||||||||||||||||||||||||||
|
והשלישית כמו ג'ד'ו' שהתוספת שיש בין ג' וד' הוא אחד ובין ד' וו' שנים [43]שערך התוספת היא כפל כערך הקטן אל הגדול | ||||||||||||||||||||||||||||||
|
|
|||||||||||||||||||||||||||||||
|
ואם ידענו השנים מהם נוכל להוציא השלישי נערך ג' על ד' עלו שנים עשר ונחסר מן הקטן התוספת שיש בינו ובין השני ועל הנשאר נחלק י"ב יצא ששה | ||||||||||||||||||||||||||||||
|
|
|||||||||||||||||||||||||||||||
|
וכן אם ידענו שהשני והשלישי ד'ו' ורצינו להוציא הקטן נערך ד' על ו' עלו כ"ד ונוסיף על הגדול התוספת שיש לו על השני ועלו שמונה ועליו נחלק כ"ד יעלה שלשה | ||||||||||||||||||||||||||||||
|
|
|||||||||||||||||||||||||||||||
|
ואם ידענו שהקטן והגדול ג'ו' ונרצה לדעת האמצעי נערוך ג' על ו' יעלו י"ח ונחבר הקטן עם הגדול יהיו תשעה ונחלק עליו שמונה עשר יצא שנים ונכפול העולה לעולם וככה האמצעי | ||||||||||||||||||||||||||||||
|
או נוסיף על הקטן רביעית הגדול ונחלק עליו והיוצא לאחד מהשלמים הוא האמצעי | ||||||||||||||||||||||||||||||
| Instructions for creating a table of arithmetic progressions based on the set of the natural numbers | |||||||||||||||||||||||||||||||
|
ואם תרצה להוציא חשבון הכפול מן האחדים תכתוב החשבון מא' ועד כמה שתרצה על סדר החשבון | ||||||||||||||||||||||||||||||
|
ואחר כך תכתוב תחתיו ותתחיל בב' ואחריו ד' שתוסיף לעולם ב'ב' וזו השטה תהיה כפל מן הראשונה על הסדר כגון שני שטין שבמכבר הראשונה והשניה | ||||||||||||||||||||||||||||||
|
ואם תרצה להוציא חשבון הכפל מן האחדים תכתוב החשבון כמוהו וחלק ממנו שהוא חצי היוצא משנים עם שלשה תכתוב הזוגות על הסדר ותתחיל משנים שהם ראש הזוגות ואחר כן תערך האחדים על הסדר על שלשה שתוסיף לעולם שלשה כמו הטור השלישי שבמכבר והוא יהיה כמוהו וחציו אל הטור השני | ||||||||||||||||||||||||||||||
|
ואם תרצה להוציא כפלו וחלק ממנו שהוא חצי היוצא מחמשה אל שנים תכתוב ב' בתחלה והוסף בב' אחריה כמו הטור השני שבמכבר ואחר כן תערוך האחדים על ה' כמו הטור החמישי שבמכבר והוא יהיה כפלו וחלק ממנו אל הטור השני | ||||||||||||||||||||||||||||||
|
ואם תרצה להוציא כמוהו וחלקים ממנו שהם שתי שלישיות היוצא מחמשה אל שלשה הוא כמו הטור החמישי שבמכבר אל הטור השלישי | ||||||||||||||||||||||||||||||
|
ואם תרצה להוציא כפלו וחלקים ממנו שהוא שתי שלישיות היוצא משמונה אל שלשה כמו הטור השמיני [44]אל הטור השלישי שבמכבר הוא | ||||||||||||||||||||||||||||||
|
וכל מספר שיש לו חלק החלק מונה אותו כמנינו או כמנין חלק אחר | ||||||||||||||||||||||||||||||
|
והמספר אשר חלקו מונה אותו כמספרו נקרא מספר מרובע מפני שצלעו האחד שוה לצלעו השני וכשאתה מונה את צלעו כמנינה תקבץ המספר הרבוע | ||||||||||||||||||||||||||||||
|
כמספר ד' וט' וי"ו וכדומה להם | ||||||||||||||||||||||||||||||
|
ואשר חלקו מונה אותו כמנין אחד מחלקיו נקרא מספר שטוח ויש לו שתי צלעות | ||||||||||||||||||||||||||||||
|
כמספר ט"ו אשר צלעו האחת ג' והשנית ה' | ||||||||||||||||||||||||||||||
|
וכשאתה מונה צלעו כמנין הצלע השנית תקבץ מספרו השטוח שהוא ט"ו | ||||||||||||||||||||||||||||||
|
וכל מספר שאתה מונה אותו באחד לבדו נקרא מספר ארוך מפני שאין לו צלע שני כי אם האחד שאינו מספר | ||||||||||||||||||||||||||||||
| All square numbers are formed successively by the sum of one, which is the first square, with the sequence of the odd numbers. | וכל המספרים המרובעים נמצאים על סדרם מקבוץ האחד אשר הוא המרובע הראשון עם המספרים הנפרדים על סדרם | ||||||||||||||||||||||||||||||
|
וענין זה אם אתה מקבץ האחד שהוא המרובע הראשון אל השלש שהוא תחלת הנפרדים אתה מוצא הרבוע השני והוא ד' אשר צלעו ב' | ||||||||||||||||||||||||||||||
|
ואם אתה מוסיף על מרובע השני המספר הנפרד השני והוא ה' יהיה הכל ט' והוא המרובע השלישי אשר צלעו ג' | ||||||||||||||||||||||||||||||
|
וכן אם אתה מוסיף על המרובע השלישי הנפרד השלישי והוא ז' יצא המרובע הרביעי והוא י"ו | ||||||||||||||||||||||||||||||
|
וכן על הסדר הזה אתה מוסיף על המרובע המספר הנפרד אשר במעלתו יצא המרובע התלוי אליו עד אין סוף | ||||||||||||||||||||||||||||||
| You find the five different kinds of ratios depend on the equivalence ratio and return to it, when you dissolve their relation, as the odd numbers create the square numbers. | וכן אתה מוצא חמשה ערכי המספר אשר הם נחלפים תלויים בערך הישר וחוזרים אליו כשאתה מתיר את קשרם כאשר המספרים הנפרדים קושרים את המרובע במספר | ||||||||||||||||||||||||||||||
| I draw for you a diagram, in which you can see the six different ratios, including the equivalence ratio, arranged with three numbers for each ratio: | והנה אני מצייר לך צורה תראה בה שש הערכים אשר הם הנחלפים עם הישר סדורים בשלשה מספרים בכל ערך וערך | ||||||||||||||||||||||||||||||
|
ואם אתה רוצה להתיר הערך הנחלף אל ישר אתה פוחת ראש מספרו מן השני וישאר בידך שלשה [45]שהם שוים או מוערכים על שרש אחד שהוא קרוב אל השוה | ||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||
|
כגון ערך החלק אשר היא המעלה השלישית בצורה הזאת ומספרנו ד' ו' ט' | ||||||||||||||||||||||||||||||
|
אם אתה פוחת ד' מן ו' ישארו בידך ב' | ||||||||||||||||||||||||||||||
|
ואם אתה פוחת מן ב' א' ואחר כך תפחות מד' שהוא המספר הגדול א' וב' ישאר לך אחד ג' פעמים והוא הערך הישר | ||||||||||||||||||||||||||||||
| All ratios return to the equivalence ratio, when you dissolve their relation and inequivalence. | והנה כל הערכים כאשר אתה מתיר את קשרם ואת חלופם חוזרים אל ערך הישר | ||||||||||||||||||||||||||||||
| The sages deduce from this an allusion about the human: if he keeps himself once in the virtues of the ascetics and restrains himself from the passions of the world, the next time will be easier for him until reaching the definition of righteousness that is derived from the image of the one that corresponds the form of the Most High; Who dwells alone; Who is everything and everything is from Him, the beginning of all and the end of all; Who knows everything in particular and in general; to Whom the spirit of everything returns; the Secret of praises and the Reason of prayers; may God's name be blessed forever and ever. | ואמרו מכאן החכמים רמז על האדם אם ישמור נפשו במעלות הפרושים ויכבוש את עצמו מתאות העולם פעם אחת יקל עליו באחרת עד שיגיע לגדר היושר היוצא מדמות האחד אשר דמות העליון כנגדו והוא שוכן לבדו והוא הכל ומאתו הכל ראשית הכל ואחרית הכל ויודע הכל בפרט וכל ואליו תשוב רוח הכל על כן סוד התהלות וטעם התפלות יהי שם יי' מבורך לעולם ועד | ||||||||||||||||||||||||||||||
Chapter Six: Deducing One from Another |
השער הששי הוצאת זה מזה |
|---|---|
In Astrology |
|
| It is known in the science of the zodiacal constellation of the seven planets. | ידוע בחכמת המזלות לשבעת המשרתים שהם כוכבי לכת |
| The mean motion of Saturn each day is approximately 2 minutes, of Jupiter 5 minutes, of Mars 32 minutes, of the Sun and Venus 29 minutes, of Mercury 3 degrees and 11 minutes, and of the Moon 13 degrees and 11 minutes. | ומהלך השוה בכל יום בדרך קרובה לשבתי ב' ראשונים ולצדק ה' ראשונים ולמאדים ל"ב ראשונים ולחמה ונוגה כ"ט ראשונים ולכוכב חמה ג' מעלות י"א ראשונים וללבנה י"ג מעלות י"א ראשונים |
|
ואם ידעת יום ידוע שנכנס אחד מן המשרתים שמהלכם במתון בראש טלה ולאחר ט"ו יום נכנס אחד מן הקלים שמהלכם במרוצה בראש ו' טלה ותרצה להוציא מתוך חשבון מהלכם בכמה ימים וכמה שעות ישיגנו ויתחבר עמו |
|
הערך המעלות או החלקים כפי מה [46]שיהיו בימים שהלך וחלק על היתרון שיש בין שתי ההליכות ומה שיצא הם ימים ומה שישאר הם חלקים מהיתרון חלק חלקי היתרון על י"ב ומה שיצא חלק חלקי היתרון עליו והם שעות וחלקי שעה |
|
ובחינתו להעריך מהלך הראשון על הימים שעברו מחוברים עם הימים שירדף אחריו השני |
|
והערך חלקי היתרון על חשבון המעלות או החלקים שילך וחלק על היתרון והם מעלות או חלקים וישארו חלקים מחלקי היתרון |
|
וכן תעשה במהלך השני שתערוך חלקי היתרון על מהלכו וחלקם על היתרון |
|
תמצאם שוים |
|
ואם ילך האחד והשני יבא כנגדו כגון שיהיה נזור ותרצה להוציא מתי יתחברו |
|
חבר מהלך שניהם והוא היום וחלק הדרך עליו |
|
ועל זה הדרך תוכל לחשב לרצים ולשלוחים אשר תדע מהלכם בלא תוספת ובלא מגרעת |
|
וגם ידוע הוא שיש למשרתים מחברות פעמים שיתחברו כלם במזל אחד ופעמים ששה מהם או ה' או ד' או ג' או ב' מהם במזל אחד ותרצה להוציא כמה הם כל המחברות שלא ידמה זה לזה |
|
וככה תוכל לדעתם דע כי כל חשבון שיחובר מאחד עד איזה מספר שתרצה תוכל להוציאו מערכו אל חצי ואל חצי אחד |
|
ונחל לספור כמה יהיו המחברות השניות והטעם שיתחברו שני כוכבים לבדם |
|
והנה יש לשבתי עם המשרתים מחברות ששה ונערוך ששה על חציו וחצי אחד יעלה כ"א וככה מספר המחברות השניות |
|
רצינו להוציא השלישיות |
|
ושמנו צדק עם שבתי ועמהם אחד מהאחרים החמשה ויעלה מספרם חמשה ערכנום על שלשה שהוא חצי המספר וחצי אחד יעלה ט"ו וזאת מחברת שבתי |
|
וראוי להיות מחברות צדק ארבעה ערכנוהו על שנים וחצי עלה עשרה |
|
ומחברות מאדים שלשה ערכנוהו על שנים עלה ששה |
|
ומחברות חמה שנים ערכנום על אחד וחצי עלה שלשה |
|
ומחברות נגה אחת |
|
והנה מספר מחברות[47] השלישיות חמשה ושלשים |
|
רצינו להוציא הרביעיות |
|
ונחל משבתי וצדק ומאדים עמו ובעבור שצריך לשלשה שיתחברו עמו תחלת המחברת ארבעה ערכנו על שנים וחצי עלו עשרה |
|
ואחר כן יהיה מחברת שבתי וצדק עם האחרים ויהיו בתחלה שלשה ערכנום על שנים עלה ששה |
|
ואחר כן יחל שבתי עם מאדים ויהיו שנים ערכנום על אחד וחצי עלה שלשה |
|
ואחרי כן מחברת אחת |
|
ועלה מספר מחברות שבתי עשרים |
|
והנה יחל צדק משלשה ערכנום על שנים עלה ששה |
|
ואחר כן שנים ערכנום על אחד וחצי עלה שלשה |
|
ואחר כן מחברת אחת |
|
והנה מחברת צדק עשר |
|
ויחל מאדים משנים ערכנום על אחד וחצי עלה שלשה |
|
ואחר כן מחברת אחת |
|
הנה ארבע מחברות למאדים |
|
ומחברת חמה עם השפלים אחת |
|
והנה מספר הרביעיות חמשה ושלשים |
|
רצינו להוציא החמישיות ומצאנו לשבתי ט"ו ולצדק ה' ולמאדים אחת והנה מספר החמישיות כ"א |
|
והמחברות הששיות יש לשבתי שש ואחת לצדק והנם שבע |
|
ומחברות השבעה אחת |
|
ועלה מספר כלם מאה ועשרים מחברות |
| In many issues the astrologers need to extract the unknown from the known: | ובעינינים רבים יצטרכו חכמי המזלות להוציא הנסתר מהידוע |
|
כמו בחשבון הקשתות והיתרים והחצים להוציא זה מזה ועם גזור האל אפרשנו במקומו בזה הספר |
|
וכאשר בחשבון צללי השמש להוציא הישר מן ההפוך וההפוך מן הישר ומן כל אחד ואחד הגובה ומן הגובה כל אחד ואחד מהם אך אין זה מקומו להאריך בו |
| Reference to Abraham Ibn Ezra's works: This is explained in Sefer Keli ha-Neḥošet [Book on the Astrolabe] and Sefer ha-Luḥot le-Šivʿah ha-Mešaretim [Tables of the Seven Planets]. | והנה מפורש בספר כלי הנחשת ובספר הלוחות לשבעה המשרתים |
In Mathematics |
|
| In the mathematicians' way you extract the unknown from the known as you have seen in the preceding chapters on addition, subtraction, multiplication, division, ratio of one to another, and we find it also in the issue of roots. By the decree of God I will explain it in its place. | ולענין חכמי המדות אתה מוציא הנסתר מן הגלוי כאשר ראית בשערים שעברו במחברת ובמגרעת [48]ובמערכת ובמחלוקת ובערך זה אל זה וגם בענין השרשים נמצאנו ועם גוזר האל במקומו אפרשנו |
| In bargaining and in human affairs you find also the extraction of the unknown from the known. | וגם בחשבוני מקח וממכר ובעסקי בני אדם אתה מוצא להוציא הנסתר מהידוע |
| For, all human affairs associated with the calculations of commerce, employment of workers, and exchange are based on four numbers in two orders of ratio [= "Rule of Three"] | כי כל עסקי בני אדם בחשבוני מקחם וממכרם ושכירות כל מעשיהם ושעור חלופיהם הם עומדים בין ארבעה מספרים בשני סדרי הערך |
| The Rule of Three is built on four numbers that are in the two orders of the equivalence ratio | |
| For every four numbers that are in the two orders of the equivalence ratio: | כי כל ארבעה מספרים בשני סדרי ערך שוה |
|
והוא שיהיה חלק האחד מן השני כחלק השלישי מן הרביעי |
|
אם אתה עורך הראשון ברביעי יהיה מספרו כמספר השני בשלישי |
|
והמשל כגון שני מספר ד' ט"ו עם שני מספרי י"ב מ"ה |
|
והראשון שהוא ד' הוא חלק מן מספר ט"ו אשר הוא השני כמו י"ב שהוא המספר השלישי מן מספר מ"ה והוא הרביעי והוא חמישיתו ושליש חמישיתו |
|
ואם אתה עורך ד' והוא הראשון במספר מ"ה והוא הרביעי יהיה מספרו כמספר ט"ו השני במספר י"ב השלישי |
|
ועוד אם תקח ערך ד' אל י"ב השלישי יהיה כערך ט"ו אל מ"ה הרביעי |
|
ואתה מוצא מספר ד' והוא הראשון נערך אל שני המספרים אל השני ואל השלישי ואינו נערך אל הרביעי |
|
וכן השני נערך אל הראשון ואל הרביעי ואינו נערך אל השלישי |
|
והמספרים הנערכים זה אל זה נקראים חברים |
|
ושאינם נערכים נקראים נכריים |
| Hence, you learn that every four numbers that are equal in ratio, if you multiply one of the ratio’s orders by its stranger from the second order, it will be equal to the product of its companion in the ratio [multiplied] by its own stranger from the other ratio.
|
ומכאן אתה למד שכל ד' מספרים שהם שוים בערך אם אתה עורך האחד מסדרי הערך עם הנכרי לו מסדר השני יהיה שוה לחשבון חברו בהקשה באשר הוא נכרי לו מן הערך השני |
Word Problems |
|
Trade |
|
| Trade is based on the "Rule of Three" | |
| All human affairs are based on the two orders of the equivalence ratio: | וכל עסקי בני אדם עומדים בשני סדרי הערך הזה |
The [amount of goods offered in] business and trade are one [term] [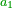 ]; the corresponding price is the second [term] [ ]; the corresponding price is the second [term] [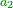 ]. ].
|
כי העסק והמסחר הוא האחד ושני לו השער המסור לו |
The second order is the taken or sold [amount of goods] [ ] and its second [term] is the money [paid for the amount of goods which was actually sold] [ ] and its second [term] is the money [paid for the amount of goods which was actually sold] [ ]. ].
|
והסדר השני הוא הנלקח או הנמכר ושני לו הדמים |
| The [amount of goods offered in] business is proportional to the price, as the sold [amount of goods] is proportional to the money [paid]. | והעסק נערך אל השער כאשר הנמכר נערך אל הדמים |
| The ratio of the [amount of goods offered in] business to the sold merchandise is equal to the ratio of the price to the money [paid] and they are the "companions". | ועוד ערך העסק אל המכר כערך השער אל הדמים ואלו הם החברים |
|
| |
| You find the [amount of goods offered in] business stranger to the money [paid] and the sold merchandise stranger to the price. | ותמצא לעולם העסק נכרי [49]לדמים והמכר נכרי לשער |
| In all human affairs three of these values are known and the fourth is unknown, but we can deduce it from the three that are known: | ובכל עסקי בני אדם שלשה מאלה הארבעה ידועים והרביעי נסתר ונוציאנו מכח השלשה הידועים |
| For, if you multiply one of the three knowns by its stranger and you get the product, then you divide the product by the third known, you find the fourth unknown. | שאם אתה עורך אחד מהשלשה הידועים בנכרי לו מהם ותדע המספר הנכלל בחשבון ותחלק אותו על השלישי הידוע אתה מוצא הרביעי הנסתר |
|
| |
| Examples | |
|
כגון האומר עשרה כורי החטה בו' דינ' כמה כורים אוכל לקחת בארבעה דינ' |
|
והמסחר הוא הי' כורים והוא הנקרא עסק |
|
והשער המסור לו הוא ו' דינ' |
|
ואלה שניהם עומדים בערך אחד |
|
והערך השני הוא הד' דינ' והוא הדמים |
|
ואתה רוצה להוציא המכר הנסתר |
|
ובזה הענין שני הנכרים אשר בשלשה הידועים הם הדמים והעסק והם י' וד' |
|
ולפיכך תערוך י' בד' ויהיו מ' |
|
חלקם על השער הידוע שהוא ו' ויהיה ששה ושני שלישי אחד והוא מספר המכר שהיה נסתר |
|
או דע מה ערך ד' שהם הדמים מן ו' שהם השער והם החברים ויהיה ב' שלישיותיו |
|
קח שני שלישיותיו העשרה שהוא העסק וככה הוא המכר שהיה נסתר |
|
או חלק העשרה על הו' והיוצא תערוך על ד' והעולה הוא המכר אשר היה נסתר |
Here, the third number [ ], which is the sold merchandise, was unknown. ], which is the sold merchandise, was unknown.
|
ובכאן היה המספר השלישי נסתר והוא המכר |
If the fourth number [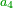 - the money paid] is unknown: - the money paid] is unknown:
|
ואם היה המספר הרביעי נסתר |
|
כגון האומר י' כורים בו' דינ' כמה הוא דמי ד' כורים |
|
ובכאן הם הדמים נסתרים |
|
ותדענו שתערוך השער שהוא ו' במכר שהוא ד' יעלה כ"ד |
|
חלקם על המסחר והוא הנקרא עסק והם הי' כורים יצא ב' דינ' וב' חומשי דינ' והוא הדמים שהיו נסתרים |
|
גם תדענו שתדרוש מה ערך הד' שהם המכר מן הי' שהם העסק והם החברים והוא שני חמישיותיו |
|
קח שני חמישיות ו' והוא הדמים הנסתרים |
|
או חלק הו' דינ' על הי' והיוצא שהם שלשה חומשין ערכם על הד' והעולה הם הדמים הנסתרים |
If the price [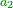 ] is unknown: ] is unknown:
|
ואם היה השער נסתר |
|
כגון האומר קניתי ג' כורים בד' דינ' בכמה הייתי קונה עשרה כורים |
|
[50]ובכאן המכר ודמיו ידועים והם ג' כורים וד' דינ' |
|
וכן המסחר שנקרא העסק ידוע והם הי' כורים |
|
ונסתר ממך שער הי' כורים |
|
ותדענו שתערוך המסחר שהם י' כורים בד' שהם דמי המכר ועלה מ' |
|
חלקם על ג' והם המכר ושא השער הנסתר והוא י"ג דינ' ושליש |
|
גם תדענו ראה מה ערך הי' מן הג' והוא כפלו ג' פעם ושליש |
|
ערכם על הד' כלו' כפול ד' ג' פעמים ושליש יעלה י"ג ושליש |
|
או חלק הד' על הג' יצא אחד ושליש |
|
ערכם על הי' יעלה י"ג ושליש |
If the [amount of goods offered in the] business [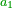 ] is unknown: ] is unknown:
|
ואם היה המסחר שהוא הנקרא עסק נסתר |
|
כגון האומר קניתי ו' כורים בד' דינ' כמה כורים אקנה בז' דינ' |
|
נמצא שאתה יודע המכר ודמיו והם ו' כורים וד' דינ' והם כערך אחד |
|
וגם אתה יודע מן הערך הראשון השער שהוא ז' דינ' |
|
ונסתר ממך העסק והוא המסחר |
|
ותדענו שתערוך השער שהם ז' בו' שהוא המכר והם הנכריים ועלו מ"ב |
|
חלקם על ד' שהם הדמים יצא המסחר שהוא העסק והוא י' כורים וחצי |
|
ותדענו שתדע מה ערך ז' אל ד' והוא כמוהו וג' רביעיותיו |
|
ערכם אל הו' והוא י' וחצי |
|
או חלק הו' על הד' יצא אחד וחצי |
|
ערכם אל על הז' יעלה י' וחצי |
| Now I have explained to you the extraction of the unknown number from the known [numbers]. | ועתה בארתי לך הוצאת המספר הנסתר מתוך הידועים |
| If you want to extract both the [amount of goods offered in the] business and the corresponding price: | ואם רצית להוציא הכורים והדמים |
|
כגון שישאל השואל ג' כורים בה' דינ' וקנה כורים נעלמים וכאשר תחבר הדמים עם הכורים יעלו ששים כמה היו הכורים והדמים |
|
חבר הג' והה' יעלה ח' |
|
ודע מה ערך הג' מן הח' והוא ג' שמיניות |
|
קח ג' שמיניות הששים והוא כ"ב וחצי והם הכורים |
|
ולהוציא הדמים דע מה ערך הה' מן הח' והוא ה' שמיניות |
|
קח ה' שמיניות הששים והם ל"ז וחצי והם הדמים |
|
או חלק הששים על שמונה יהיה היוצא ז' וחצי |
|
אם תערכם על הג' יעלה חשבון הכורים |
|
[51]ואם תערכם על ה' יעלה חשבון הדמים |
|
ואם תרצה להוציא ג' כורים בז' דינ' וקנה כורים נעלמים כשתגרע מהם הדמים ישאר כ' כמה היו הכורים והדמים |
|
גרע הג' מן הז' ישאר ד' |
|
ודע מה ערך הג' מן הד' והוא ג' רביעיות |
|
קח ג' רביעיות הכ' והוא ט"ו והם הכורים |
|
ולהוציא הדמים דע מה ערך הז' מן הד' והוא כמוהו וג' רביעיותיו |
|
קח כערכם מן הכ' והוא ל"ה והם הדמים |
|
או אם תחלק הכ' על ד' יצא ה' |
|
אם תערכם על ג' יעלה חשבון הכורים |
|
ואם תערכם בז' יעלה חשבון הדמים |
|
ואם תוציא ג' כורים בה' דינ' וקנה כורים נעלמים כשתערוך הכורים על הדמים יעלה ששים |
|
ערוך הג' בה' יעלה ט"ו |
|
וחלק עליהם הששים ומהעולה קח השורש והוא ב' |
|
וערכם על ג' יהיה ששה והם הכורים |
|
ואם תערוך הב' על הה' יעלה י' והם הדמים |
|
או אם תערוך הג' בששים יצא ק"פ |
|
אם תחלקם על הה' יצא ל"ו |
|
ומהיוצא תקח השרש והעולה הוא חשבון הכורים |
|
ואם תחלקם על הג' ומהיוצא תקח שרש והעולה הוא חשבון הדמים |
|
ואם תוציא ד' כורים בט' דינ' וקנה כורים נעלמים אם תחבר שורש הכורים עם שורש הדמים יעלה ז' וחצי |
|
קח שורש הד' והוא ב' |
|
וקח שורש הט' והוא ג' |
|
חבר ב' עם ג' יהיה ה' |
|
חלק עליהם הז' וחצי והיוצא תערוך בשנים יעלה ג' והם שורש הכורים ומספרם ט' |
|
ואם תערוך היוצא בחלוק בג' יהיה ד' וחצי והם שורש הדמים |
|
תערכם על עצמם יעלה כ' דינ' ורובע דינ' |
|
או אם תחלק הט' על הד' יצא ב' ורובע |
|
קח השורש והוא אחד וחצי |
|
הוסף עליו אחד לעולם יהיה שנים וחצי |
|
חלק עליהם הז' וחצי והיוצא שהם ג' תערוך [52]על עצמם והעולה הוא חשבון הכורים |
|
| |
|
ולדעת הדמים תחלק הד' על הט' יצא ד' תשיעיות |
|
קח השרש והוא ב' שלישיות שהם שורש ד' תשיעיות |
|
הוסף עליהם אחד יהיה אחד וב' שלישיות |
|
חלק עליהם הז' וחצי יצא לאחד שלם ד' וחצי |
|
ערכם על עצמם והוא חשבון הדמים |
|
ואם תוציא ד' כורים בט' דינ' כשתפחות שרשו משורש הדמים ישאר אחד וחצי |
|
קח שורש הד' והוא ב' |
|
וחסרם משרש הט' ישאר א' |
|
חלק עליו אחד וחצי יצא הכל |
|
אם תערכנו עם שנים יעלה שורש הכורים |
|
ואם תערכנו על שלשה יצא שרש הדמים |
|
ותוכל להוציאו בדרך האחר |
|
ואם תוציא ד' כורים בט' דינ' כשתערוך שורש הכורים על שורש הדמים יהיה כ"ד |
|
קח שרש הד' והוא ב' |
|
ערכם בגדר הט' יעלה ששה |
|
חלק עליהם הכ"ד והיוצא תח תערוך בד' יהיה י"ו והוא חשבון הכורים |
|
ואם תערוך היוצא בחלוק על ט' יעלה ל"ו והוא חשבון הדמים |
|
ואם תוציא כורים נעלמים ודמיהם ששים ודמי הכור כשיתחברו עם חשבון הכורים יהיה י"ו |
|
קח חצי הי"ו והוא ח' |
|
ערכם על עצמם ומהעולה חסר הששים ישאר ארבעה |
|
קח השורש והוא ב' |
|
והוסף על הח' יהיו י' והם הכורים |
|
| |
|
וחסר הב' מן הח' ישאר ו' והם הדמים |
|
ואם תוציא כורים נעלמים ודמיהם ששים אם תחסר דמי הכור מחשבון הכורים ישאר ארבעה |
|
קח חצי הד' ותערכנו על עצמו יהיה ד' |
|
חברם עם הששים וקח השרשים והוא ח' |
|
הוסף עליהם השנים והוא י' והם הכורים |
|
או אם תחסר הב' מן הח' ישאר ששה והם דמי הכור |
|
ואם תוציא כור בג' וכור בז' ורוצה לקנות משניהם כור בו' כמה יקח מכל אחד ואחד |
|
דע מה [53]בין הג' והז' והוא ד' |
|
ואחר כך תגרע הו' מן הז' ישאר אחד |
|
ודע מה ערכו מן הד' והוא רביע וככה יקח מן הכור שהוא בג' רביע |
|
ומותר הכור שהם ג' רביעיות יקח מן הכור שהוא בז' |
|
ואם תוציא כור בג' וכור בד' וכור בה' ורוצה ליקח בב' דינ' מכל כור וכור בשוה כמה יקח מכל אחד |
|
חבר הג' והד' והה' יהיו י"ב |
|
ודע מה |
|
ואם תוציא כור בג' וכור בד' וכור בה' ולקח משלשתם כור וחצי מכל אחד בשוה כמה הם הדמים |
|
חבר הג' והד' והה' יהיו י"ב |
|
ודע מה ערך הכור וחצי מן הג' כורים וכערכם קח מן הי"ב והוא ו' והם הדמים |
|
ואם תוציא כור בג' וכור בז' וכור בי"ב וירצה לקח כור בי' דינ' כמה יקח מכל מין ומין |
|
חבר הג' והז' והוא עשרה ושמרם |
|
ואחר כך כפול הי"ב יהיו כ"ד |
|
וחסר מהם הי' ששמרת ישאר י"ד |
|
ואחר חסר הי' אשר לקח מהם הכור מן הי"ב ישאר שנים |
|
ודע מה ערכם מן הי"ד והוא שביעית וככה קח מן הכור שהוא בג' וכמו כן מן הכור שהוא בז' |
|
והנשאר שהוא ה' שביעיות נקח מן הכור שהוא בי"ב |
|
ואם תוציא כור בב' דינ' וכור בג' וכור בה' וכור בי"ד ורוצה לקח כור בי"ב דינ' כמה יקח מכל מין ומין |
|
חבר הב' והג' וה' יעלו עשרה |
|
ואחר כך כ"כ כפול הי"ד שלשה פעמים לפי שעלו הדמים בדמי ג' המינין אך למעלה לא כפלנוהו אלא פעם אחת לפי שלא עלו הדמים לדמי ג' המינין וכשכפלנו הי"ד ג' פעם עלה מ"ב |
|
חסר מהם הי' ישאר ל"ב |
|
ואחר חסר הי"ב מן הי"ד ישאר שנים |
|
ודע מה ערכם מן הל"ב והוא חצי שמינית וככה יקח מן הכור שהוא בב' דינ' וכן מן הג' וכן מן הה' |
|
והנשאר שהוא ו' שמיניות וחצי שמינית יקח מן הכור שהוא בי"ד |
|
ואם תוציא עשרה כורים בעשרים ושנים עשר בעשרים וחמשה עשר כורים בעשרים וירצה לקח בעשרים חלק שוה מכל מין ומין כמה יתן לו |
|
חלק העשרים [54]על עשרה יצא שנים ועל י"ב יצא אחד ושני שלישיות ועל ט"ו יצא אחד ושליש |
|
חבר הכל יהיו חמשה |
|
חלק עליהם העשרים יצא ארבעה וכן יקח מכל מין ומין |
|
ואם תוציא ד' כורים בח' זוז פחות דבר שאינו ידוע ולקח שני כורים בשאינו ידוע וזוז כמה היה מה שאינו ידוע |
|
הוסף הב' כורים על הד' יהיו ו' |
|
ואחר כך חבר שאינו ידוע וזוז עם הח' פחות דבר שאינו ידוע יהיו תשעה |
|
ודע מה ערך שני הכורים מן הששה והוא שליש |
|
קח שליש התשעה וחסר ממנו הזוז והנשאר שהוא שני זוזים הוא הדבר שאינו ידוע |
|
| |
|
ואם תוציא חמשה כורים בעשרה דינ' פחות משהו ולקח ג' כורים במשהו שאינו ידוע פחות דינ' עם כמה זה המשהו |
|
חבר הג' עם הה' יהיו ח' |
|
ואחר כך חבר המשהו פחות דינ' עם הי' פחות משהו יהיה ט' דינ' |
|
ודע מה ערך הג' כורים מן השמונה והוא רביעית וחצי רביעית |
|
וכערכם קח מן הט' והוא ג' ושלשה שמיניות |
|
הוסף עליהם הדינר הפחות והם ד' וג' שמיניות והוא המשהו |
|
| |
Employment of workers |
|
| Likewise you find in the employment issue. | וכן אתה מוצא בענין השכירות |
|
כגון האומר שכרתי פועל לשלשים יום בי' דינ' ועשה על ח' ימים כמה שכרו |
|
ובכאן אתה חושב ל' ימי השכירות למסחר |
|
והי' דינ' לשער |
|
וח' ימים שעשה מלאכתו למכר |
|
ויהיה המספר הנסתר דמי המכר |
|
ותוציאנו שתערך הח' בי' יהיו פ' |
|
חלקם על הל' יצא ג' פחות שליש |
|
ותדענו שתדע מה ערך ח' אל ל' והוא ד' חמשיות שלישית וכערכם קח מן הי' יצא ג' פחות שליש |
|
ותדענו שתחלק הי' על הל' יצא שליש |
|
ערכהו על ח' יצא והוא ח' שלישיות והדבר שוה |
| With these methods you solve when the sold merchandise, or price, or [amount of goods offered in the] business are unknown. There is no need to elaborate. | ועל אלו הדרכים תוציא כשיהיו המכר או השער או המסחר נסתרים ואין צריך להאריך בזה |
|
ואם תוציא שכיר בימים נעלמים בדינר נעלמים וכשתחבר הימים והדינ' יהיו מ' ועשה ימים נעלמים כשתערכם בשכרם יעלה י"ב ואם תערך הנשאר [55]מן הימים בנשאר מן השכר יעלה קצ"ב כמה הם הימים הנעלמים וכמה הדינ' וכמה עשה מן הימים |
|
|
|
|
|
|
|
חלק הקצ"ב על י"ב יצא י"ו |
|
קח שרשם והוא ד' והוסף עליהם אחד יהיה ה' |
|
וחלק עליהם המ' יצא ח' |
|
קח חצים והוא ד' וערכם על עצמם ומהעולה חסר הי"ב ישאר ד' |
|
קח שרשם והוא ב' הוסיפם על הד' שהם חצי הח' והעולה שהוא ו' הם הימים הנעלמים אשר עשה |
|
| |
|
|
|
|
או דע מה ערך הי"ב מן הק'צ'ב' והוא חצי שמינית קח השרש והוא רובע הוסף עליו אחד לעולם יהיה אחד ורובע |
|
חלק עליהם המ' יצא ל"ב |
|
קח חצים והוא י"ו וערכם על עצמם יעלה רנ"ו |
|
חסר מהם הק'צ'ב' יהיה הנשאר ס"ד |
|
קח שרשם והוא ח' הוסיפם על הי"ו יהיו כ"ד |
|
חברם עם הו' ראשונים והמחובר שהוא ל' הם הימים הנעלמים מן השכירות |
|
| |
|
וחסרם מן המ' והנשאר שהוא י' הם הדינ' הנעלמים |
|
ואם תוציא שכיר בחדש בי' דינ' ואם יבטל ישלם חצי שכרו ועשה ובטל ויצא שכרו בהפסדו |
|
חבר הה' שהוא חצי השכר עם הי' יהיה ט"ו |
|
ודע מה ערך הה' מהם והוא שליש וכערכם קח ממי החדש והיוצא שהוא י' הוא הימים שעשה |
|
ובטל שאר הימים והם כ' |
|
או תחלק הי' דינ' על הה' דינ' יצא ב' |
|
הוסף עליהם אחד לעולם יהיה ג' |
|
וחלק עליהם ימי החדש והיוצא שהוא י' הם הימים שעשה |
|
ואם תוציא שכיר בחדש בי' דינ' ואם יבטל ישלם ה' דינ' בחדש ועשה ובטל והיה שכרו זוזים נעלמים |
|
חבר הה' עם הי' יהיו ט"ו |
|
הוסף הזוזים על הה' יהיו ו' |
|
גרע ודע מה ערכם מן הט"ו וכערכם קח מימי החדש והוא י"ב והם הימים שעשה |
|
ובטל הנשאר והם י"ח |
|
ולקח בשכרו שכר ב' ימים |
|
או חלק הי' על ה' והיוצא שהוא ב' הוסף עליהם אחד לעולם ויהיה ג' |
|
ודע מה ערך הזוזים מן הה' והוא חומש |
|
הוסיפם על ימי החדש חמישיתם יהיה ל"ו |
|
חלקם על הג' והיוצא הם הימים שעשה |
|
ואם תוציא שכיר בחדש בי' דינ' ואם יבטל ישלם ה' דינ' בחדש ועשה ובטל ונתחייב לשלם זוזים נעלמים |
|
[56]חבר הה' עם הי' יהיה ט"ו |
|
ואחר כן חסר דינ' מן הה' ישאר ד' |
|
ודע מה ערכם מן הט"ו וכערכם קח מימי החדש והוא ח' והם הימים שעשה |
|
ובטל כ"ב יום ונתחייב לשלם שכר ב' ימים |
|
או חלק הי' על הה' יצא ב' |
|
הוסף עליהם אחד יהיה ג' ושמרם |
|
ואחר דע מה ערך דינ' מן הה' דינ' והוא חמשים |
|
וקח מימי החדש חמישיתם ישאר כ"ד |
|
חלקם על הג' ששמרת והיוצא שהוא ח' הם הימים שעשה |
|
ואם תוציא שכיר בחדש בדינ' נעלמים ואם יבטל ישלם ה' דינ' בחדש ועשה ימים נעלמים וכשתערוך הימים הנעלמים על הדינ' הנעלמים היה פ' וכשיצא ממלאכתו נתחייב דינ' נעלמים |
|
חסר הדינר מן הה' ישארו ד' |
|
ערכם על הל' ומהמחובר חסר פ' ישאר מ' |
|
חלקם על ה' והיוצא שהוא הם הימים שעשה |
|
חלק עליהם הפ' והיוצא שהוא י' הם הדינ' הנעלמים |
|
ונתחייב לשלם שכר ב' ימים |
|
ואם תערוך הדינ' בל' ותחבר העולה עם הפ' יהיה ק"י |
|
חלקם על הה' והיוצא שהוא כ"ב הם ימי הבטלה |
|
ואם תוציא שכר ג' פועלים אחד ג' דינ' בחדש והשני ו' דינ' והשלישי י' דינ' ועשו בין שלשתם חדש אחד ולקחו כל אחד בשוה כמה עשה כל אחד מהם |
|
דע מה ערך הג' מן הי' והוא ג' עשיריות |
|
ודע מה ערך הג' מן הו' והוא חצי |
|
חברהו אל הג' עשיריות יהיה ד' חומשין |
|
הוסף עליהם אחד לעולם יהיה אחד וד' חומשין |
|
ודע מה האחד מהם והוא חמשה תשעיות |
|
קח חמש תשעיות ימי החדש שהם י"ו ושני שלישי יום והם הימים שעשה הראשון |
|
| |
|
וקח חצי מלאכת הראשון והוא ח' ושליש והם הימים שעשה השני |
|
או חלק הל' על הג' ודע מה ערך הל' מי"ח והוא כמוהו ושני שלישיותיו |
|
וכערך הזה קח מכל מה שיצא בחלוק לכל אחד ואחד והם הימים שעשה כל אחד ואחד |
|
ואם תוציא שכר ג' פועלים האחד ל' יום בדבר שאינו ידוע והשני בחצי הדבר שאינו ידוע והשלישי [57]בשלישית הדבר שאינו ידוע ועשו ל' יום בין שלשתם ולקח הראשון ב' דינ' והשני ג' די' והשלישי ד' די' כמה היה הדבר שאינו ידוע וכמה עשה כל אחד |
|
ערוך חלק הראשון בימי החדש יעלה ס' |
|
וערוך חלק השני בימי החדש והעולה תכפול ג' פעם יעלה ש"ס |
|
חבר הכל יהיה ת"ר |
|
חלקם על ימי החדש והיוצא שהוא כ' הוא הדבר שאינו ידוע |
|
| |
|
ולדעת הימים שעשה הראשון דע מה ערך ס' מת"ר והוא עשירית |
|
וכערכו קח מימי החדש והם ג' והם הימים שעשה הראשון |
|
ודע מה ערך הק"פ מת"ר וכערכם קח מימי החדש[58] והם ט' ימים שעשה השני |
|
ודע מה ערך הש"ס מת"ר והוא ג' חמישיותיו |
|
וכערכם קח מימי החדש והם י"ח ימים שעשה השלישי |
|
ואם תוציא שם שכיר בו' דינ' ובדבר שאינו ידוע חדש אחד ועשה עשרה ימים ולקח הדבר שאינו ידוע כמה הוא |
|
ערוך ימי החדש בו' דינ' עלה ק"פ ושמרם |
|
ואחר כן חסר י' ימים שעשה מימי החדש והנשאר שהם כ' הוא המורה |
|
וחלק ק"פ ששמרת על המורה יצא ט' והוא הכל הידוע |
|
ושאינו ידוע חסר הידוע ישאר מה שאינו ידוע והוא ג' |
|
דרך אחרת חסר הימים שעשה מימי החדש והנשאר שהוא כ' הוא המורה |
|
ואחר כן ערוך הימים שעשה על הו' דינ' יעלה ס' |
|
חלקם על המורה יצא ג' והוא הדבר שאינו ידוע |
|
דרך אחרת דע מה ערך הימים שעשה אל המורה והוא חצי |
|
וכערך זה קח מן הו' דינ' הידוע והוא ג' והם הדבר שאינו ידוע |
|
ואם היה חשבון שלא היה לו ערך |
|
ערוך הימים שעשה על הו' דינ' והעולה חלק על המורה אז תמצא הדבר שאינו ידוע |
|
ואם אמר ולקח בשכרו הדבר שאינו ידוע ונתן לו עוד דינר |
|
הוסף על הדבר הידוע אחד ועשה כמשפט |
Partnership |
|
| You also find two orders of ratio in the issue of partnership: | וכן לענין שתוף אתה מוצא שני סדרי הערך |
For, all the parts of the money that are contributed to the partnership are as [the amount of goods offered in] business, which is the trade [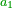 ]. ].
|
כי כל ראשי הממון שמכניסים בשתוף הם כעין העסק שהוא [59]המסחר |
The total profit is as the corresponding price. [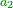 ]. ].
|
וכל הריוח הוא כעין השער המסור לו |
The share of each [of the partners] in the capital is as the sold [amount of goods] [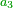 ]. ].
|
וחלק כל אחד מהממון כעין המכר |
And the part owed to each [of the partners] from the profit is as the money paid [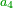 ]. ].
|
והחלק המגיע לכל אחד מן הריוח כעין הדמים |
| If the money paid [= the part owed to each of the partners from the profit] is unknown: | ואם הדמים הנסתרים |
|
כגון שנשתתפו האחד בי' והשני בכ' והשלישי בל' והרויחו ל' |
|
ערוך השער על המכר וחלק על העסק יצא חלק |
| If the sold merchandise [= share of each of the partners in the money] is unknown: | ואם המכר נסתר |
|
כגון שנשתתפו בין כלם בששים ורוחו ל' ולקח האחד ה' והשני י' והשלישי ט"ו |
|
ערוך המסחר בדמי האחד וחלק על השער יצא חלקו |
| If the price [= total profit] is unknown: | ואם השער נסתר |
|
כגון האחד י' והשני כ' והשלישי ל' ורוח בעל הי' חמשה |
|
ערוך העסק בדמי המכר וחלק על המכר |
| If the business [= all the parts of the money given to the partnership] is unknown: | ואם העסק נסתר |
|
כגון שידוע שחלק האחד היה י' ורוחו בין כלם ל' ולקח בעל הי' חמשה מן הריוח |
|
ערוך השער על המכר וחלק על הדמים |
| In all the other ways you can extract them also as it is in employment and trade. | וגם בשאר כל הדרכים תוכל להוציאם כאשר הם בשכיר ובמקח וממכר |
|
וכן אם היו ד' שותפים לכל אחד ראש ממון שאינו שוה לחברו [כלו' ה' וי' וט"ו וכ'] ויצא להם ריוח |
|
הערך ראש ממון כל אחד בפני עצמו בריוח וחלק על המחובר מראש ממון כלם שהוא נ' והיוצא הוא בחלק חלקו המגיעו מן הריוח |
|
ואם ישאר שלא יתחלק השב הדי' לפשיטים וחלק על מה שחלקת |
|
או חלק המחובר שהוא נ' על החלק ועל היוצא שהוא י' תחלק הריוח |
|
ובדרך אחרת תוכל לדעת כמה חלק כל אחד מן הריוח |
|
שתחבר ראש ממונם ודע מה ערך הריוח מראש ממונם וכערכו קח מראש כל אחד והוא חלקו |
|
ותדענו שתחלק ראש ממון הגדול על הקטן ושמור היוצא |
|
ועוד חלק ראש ממון האמצעי על הקטן והיוצא הוא חלק הראשון |
|
וראה מה ערך ראש ממון הקטן אל האמצעי וכערכו תוסיף על חלקו מן הריוח והוא חלק האמצעי |
|
וכן תוסיף לשלישי כערכו אל הקטן |
|
ואם תרצה להוציא ג' נשתתפו האחד כ' והשני ל' והשלישי מ' והריוח בעל הכ' ג' כמה הגיע לחלק האחרים |
|
חלק הל' על הכ' והיוצא תערכנו בג' שרוח והעולה [60]הוא חלק בעל הל' והוא ד' וחצי |
|
וכן תחלק המ' על הכ' והיוצא תערוך בג' והעולה שהוא ו' הוא חלקו |
|
ותדענו שתדע מה ערך הג' אל הכ' והוא ג' רביעיות חמישית |
|
וכערכו קח מראש ממון השני והשלישי |
|
ואם תוציא האחד כ' והשני ל' והשלישי מ' וריוח בעל הכ' כשתחברנו אל ריוח בעל הל' יעלה עשרה |
|
חבר הכ' והל' יהיו חמשים |
|
חלק עליהם הי' יצא חומש |
|
קח חומש ראש ממון כל אחד |
|
יקח בעל הכ' ד' |
|
ובעל הל' ו' |
|
ובעל המ' ח' |
|
ואם תוציא האחד י' והשני ל' והשלישי נ' וכשתחבר ריוח הראשון והשני ותחסרם מריוח השלישי ישאר ג' |
|
חבר הי' והל' יהיו מ' |
|
ותגרעם מן הנ' ישאר י' |
|
ודע מה ערך ג' מי' והוא ג' עשיריות |
|
קח ג' עשיריות ראש ממון כל אחד וככה חלקו |
|
הראשון ג' |
|
והשני ט' |
|
והשלישי ט"ו |
|
ואם תוציא האחד י' והשני כ' והשלישי מ' וכשתערוך ריוח הראשון והשני בריוח השלישי עלה מ"ח כמה היה ריוח כל אחד |
|
חבר הי' עם הכ' והעולה תערוך על המ' יעלה אלף ומאתים |
|
דע מה ערך המ"ח מהם והוא חמישית החומש |
|
חשוב אותם כאלו הם שברים וקח השרש והוא חומש |
|
וקח חומש ראש ממון כל אחד מהם |
|
יקח הראשון ב' |
|
והשני ד' |
|
והשלישי ח' |
|
ואם תוציא שני שותפין האחד ח' והשני י"ח ורוחו וכשתערוך שורש ריוח האחד בשורש ריוח השני יהיה ו' |
|
ערוך הו' על עצמם יעלה ל"ו |
|
ואחר כן ערוך הח' על הי"ח יעלה קמ"ד |
|
חלק עליהם הל"ו והיוצא שהוא רוביע קח שרשו והוא חצי |
|
אם תערכהו על השמונה יעלה ד' והוא הריוח של בעל הח' |
|
ואם תערכהו על י"ח יעלה ט' והוא הריוח של בעל הי"ח |
|
ותדענו שתחלק הי"ח על הח' יצא ב' ורביע |
|
קח שרשם והוא אחד וחצי |
|
חלק עליו הו' והיוצא שהוא ד' הוא ריוח הראשון |
|
ואם תערוך הד' בשנים ורובע יעלה ט' והוא ריוח השני |
|
ואם תוציא שני אנשים נשתתפו זה בה' ככר וזה בג' ככר ונשתתף עמהם השלישי שלא הביא [61]כלום והוציאו שלשתם אותם הח' ככר ושלם להם השלישי ח' ליטרין כסף כמה יקח כל אחד |
|
קח שליש הח' ככר והוא ב' וב' שלישיים |
|
חסרם מן הה' ישארו ב' ושליש |
|
ודע מה ערכם מן הב' וב' שלישיים והוא ז' שמיניות |
|
קח ז' שמיניות הח' שהוא והוא יהיה חלק בעל הה' ככר |
|
ואם תחסר הב' וב' שלישים מן הג' ישאר שליש |
|
ותדע ערכו אל ב' ושליש והוא שמינית |
|
וקח שמינית הח' והוא אחד והוא חלק בעל הג' ככרים |
|
ואם תרצה תוציא ג' אנשים נשתתפו האחד בט' ככר והשני בח' והשלישי בז' ונשתתף עמהם הרביעי שלא הביא כלום והוציאו בין שלשתם הכל ושלם הרביעי להם י"ח ליט' כסף כמה יקח כל אחד |
|
חלק כל הככרים על הד' אנשים יצאו ו' |
|
חסרם מן הט' ישאר ג' |
|
ומן הח' ישאר ב' |
|
ומן הז' ישאר אחד |
|
חבר כל השיורין וחלק עליהם הי"ח יצא ג' |
|
אם תערכם בג' יעלה ט' והוא חלק בעל הט' |
|
ואם תערכנו בב' יעלה ו' והוא חלק בעל הח' |
|
ואם תערכהו באחד יהיה ג' והוא חלק בעל הז' |
|
ואם תוציא שלשה נשתתפו בראש ממון שוה כל אחד לחבירו ומה שהביא האחד היה ארבע חומשי ממונו והשני הביא חמש שביעיות ממונו והשלישי שש תשיעיות ממונו כמה היה לכל אחד ואחד |
|
הערך השנים הראשונים זה על זה באלכסון והטעם שתערוך שברי האחד על חשבון חברו ותחשבם כאלו הם שלמים |
|
והנה הראשון כ"ה |
|
והשני כ"ח |
|
ועשה כן לשני גם לשלישי |
|
והנה השני מ"ב |
|
והשלישי מ"ה |
|
ודע כמה הוסיף השני על הראשון והוא שלש רביעיות שביעית |
|
חסר כן ממ"ב ישארו ל"ז וחצי והוא ראש ממון הראשון |
|
וד' חמשיותיו ל' |
|
וראש ממון השני מ"ב |
|
וה' שביעיותיו ל' |
|
וראש ממון השלישי [62]מ"ה |
|
ושש תשיעיותיו ל' |
Coinage |
|
| You also find two orders of ratio in the issue of coinage: | וגם בענין קשור המטבע אתה מוצא שני סדרי ההקשה |
| For the liṭra is a kind of [goods offered in the] business. | כי הליטרא כעין המסחר |
| The silver [in it] is a kind of its price. | והכסף הראוי לה כעין השער |
| The ՚oqya are a kind of a sold merchandise. | והאוקיאות כעין המכר |
| The silver in the ՚oqya is a kind of money [paid]. | והכסף המגיע לאוקיא כעין הדמים |
|
ואם היו הדמים נסתרים |
|
כגון האומר ליט' מטבעות שהיא י"ב אוקיאות ויש בהם ד' אוקיאות כסף כמה כסף יש בג' אוקיאות |
|
ערוך השער במכר וחלק על המסחר |
|
ואם היה המכר נסתר |
|
כגון האומר ליט' בד' אוקיאות כסף בכמה יש אוקיא מכסף |
|
ערוך המסחר בדמים וחלק על השער |
|
ואם היה השער נסתר |
|
כגון האומר קניתי ג' אוקיאות באוקיא כסף בכמה אקנה הליטרא |
|
ערוך המסחר בדמים וחלק על המכר |
|
ואם היה המסחר נסתר |
|
כגון האומר קניתי ג' אוקיאות מטבעות באוקיא כסף כמה אקח מן המטבעות בד' אוקיא כסף |
|
ערוך השער במכר וחלק על הדמים |
|
ואם תרצה לקשור מטבע מא' אוקיאות כסף בליט' והשני מב' אוקיאות כסף בליט' והשלישי מו' אוקי' בליטרא ואתה צריך לדעת כמה תקח מכל מטבע ומטבע ויהיה המחובר לד' אוק' אוקיאות בליט' |
|
ככה תדענו שתחבר הכסף שיש בשני המטבעות הפחותות ויעלה שלשה |
|
ואחר תכפול המטבע השלישי ויהיה הכפול י"ב |
|
וגרע מהם העולה מהמחובר משני המטבעות ישאר ט' ושמרהו |
|
ואחר תגרע הד' שהוא רוצה לקשור מן הששה שהוא סך המטבע השלישי ישאר ב' |
|
ודע מה ערך זה הנשאר אל הנשאר השמור והוא ב' תשיעיות וככה תקח מכל אחד ואחד משני המטבעות |
|
ויעלה בין שניהם ה' אוקיאות ושליש |
|
ויש בהן כסף ב' שלישי אוקיא |
|
ומן המטבע השלישי יקח ו' אוקי' וב' שלישי אוקי' |
|
שיש בהן כסף ג' אוקי' ושליש אוקי' |
|
ותעלה בידך ליט' שיש בה ארבע אוקיאו' כסף |
|
ואם יש בידך מן המטבעות ליט' באוקי' כסף וליט' בה' אוקי' וליט' בז' אוקי' ותרצה לקשור ליט' בד' אוקי' כסף |
|
[63]כן תעשה חבר שני המטבעות הגדולות מן המטבעות שהוא רוצה לקשור ויעלה י"ב |
|
וכפול השלישי ויעלה ב' |
|
וגרע אותו מהעולה מהמחובר ישאר י' ושמרהו |
|
ואחר גרע האחד שהוא המטבע היחידי מן הד' שהוא רוצה לקשור ישאר ג' |
|
ודע מה ערך זה הנשאר אל הנשאר השמור והוא ג' עשיריותיו וככה יקח מכל אחד ואחד משני המטבע |
|
ויעלה בין שניהם שבעה אוקי' וחומש אוקי' |
|
יש בהם כסף ג' אוקיאות וחצי וחצי חומש |
|
ומן המטבע השלישי ד' אוקיאות וד' חומשין |
|
יש בהם כסף ב' חומשין |
|
והנה מה שרצינו |
|
ואם יש בידך ליט' בג' רביעיות אוקי' וליטר' באוקיא ורביע וליט' בו' אוקיאו' ותרצה לקשור בד' אוקי' בליט' |
|
ככה תדענו חבר שני המטבע הפחותות יהיה ב' |
|
וכפול השלישי ויעלה י"ב |
|
וגרע ממנו המחובר ישאר י' ושמרהו |
|
ואחר גרע הד' מן הו' ישאר ב' |
|
ודע ערכם מהנשאר השמור והוא חומש וככה תקח מכל אחד ואחד משני המטבעות שחברת |
|
ויעלה בין שניהם ארבעה וד' חמישיות |
|
ויש בהם כסף ב' חומשין |
|
ומהמטבע השלישי ז' וחומש |
|
יש בהם כסף ג' וחצי וחצי חומש |
|
והנה מה שרצינו |
|
ואם יש בידך ליט' בשני אוקי' וליט' בה' ורביע וליט' בו' וג' רביעיות וקושר בד' בליטר' |
|
ככה תדענו חבר השנים הגדולים יעלה י"ב |
|
וכפול השלישי יעלה ד' |
|
וגרע הכפול מהמחובר ישאר ח' ושמרם |
|
ואחר כן גרע הב' מן הד' ישאר ב' |
|
וערכם אל הנשאר השמור והם רביע וככה יקח מכל אחד ואחד משני המטבע |
|
ויעלה בין שניהם ו' אוקי' |
|
ויש בהם כסף ג' אוקי' |
|
ומן השלישי יקח ו' אוקי' |
|
יש בהם כסף אוקי' |
|
והנה מה שרצינו |
|
ואם יש בידך ליט' ברביע אוקי' וליט' בחצי אוקי' וליט' באוקי' ורביע וליט' בה' אוקי' וקושר בד' אוקי' בליט' |
|
ככה תעשה חבר הג' מטבע הפחותות ויעלה ב' |
|
וכפול השלישי ג' פעמים בעבור שחברת ג' מטבעו' ויעלה [64]ט"ו |
|
וגרע המחובר מהכפול ישאר י"ג ושמרם |
|
ואחר כך גרע הד' מן ה' ישאר אחד |
|
וערכו מהנשאר השמור חלק אחד מי"ג וככה יקח מכל אחד מהשלשה המטבעו' המחוברות |
|
ויעלה בין שלשתם ב' אוקי' ועשרה חלקים מי"ג באחד |
|
ויש בהם כסף ב' חלקים מי"ג באחד |
|
ומן השלישי יקח ט' אוקי' וג' חלקים מי"ג |
|
יש בהם כסף ג' אוקי' ואחד עשר חלקים מי"ג באחד |
|
ונתברר מה שרצינו |
|
ואם יש בידך ליט' בג' אוקי' וליט' בחמשה ורביע וליט' בששה וחצי ליט' בז' ורביע וקושר בד' בליט' |
|
ככה תעשה חבר הג' מטבעו' הגדולות ויעלו י"ט |
|
וכפול השלישי ג' פעם ויעלה ט' |
|
וגרע הכפול מהמחובר ישאר י' ושמרם |
|
ואחר גרע הג' מן הד' ישאר אחד |
|
וערכו אל הנשאר השמור עשירית וככה יקח מכל אחד ואחד מהשלשה המחוברות |
|
ויעלה בין שלשתם ג' אוקי' וג' חומשין |
|
ויש בהם כסף ב' אוקי' וחצי חומש |
|
והנה מה שרצינו |
| ואם יש בידך ליט' בב' אוקי' | |
|
ואם יש בידך ליט' בחצי אוקי' וליט' בג' רביעיות וליט' בב' וחצי וליט' בג' ורביע וליט' בה' וג' רביעיות וקושר בג' וג' רביעיות |
|
| |
|
ככה תדענו חבר הד' מטבעו' הפחותות ויעלה ז' |
|
וכפול החמישי ד' פעם ויעלה כ"ג |
|
וגרע המחובר מהכפול ישאר י"ו ושמרם |
|
ואחר חסר הג' וג' רביעיות מן הה' וג' רביעיות ישאר ב' |
|
וערכם אל הנשאר השמור והם שמינית וכן יקח מכל אחד מהד' מטבעו' |
|
| |
|
ויעלה מארבעתם ו' אוקי' |
|
ויש בהם כסף [65]ז' שמיניות אחד |
|
ומן החמישי יקח ו' אוקי' |
|
ויש בהם כסף ב' אוקי' וז' שמיניות |
|
וזה שרצינו |
|
ואם הוא רוצה לקשור בד' אוקי' בליט' ויש מטבעות שכלם פחותות מד' כגון שיש בידו ליט' מג' רביעיות אוקי' כסף בליט' וליט' באוקי' ורביע וליט' באוקי' וחצי וליט' בב' אוקי' וחצי ורוצה להתיך מכלם בשוה וצריך לערב בהם כסף |
|
| |
|
ככה תדענו חבר הד' מטבעו' ויעלה ששה |
|
וכפול ליט' של כסף ד' פעם יעלה מ"ח אוקי' |
|
חסר המחובר מהכפול ישאר מ"ב ושמרם |
|
ואחר כך חסר הד' מן הליט' כסף ישאר ח' אוקי' |
|
וערכם מהנשאר השמור שביעית ושליש שביעית וככה יקח מכל אחד מהם |
|
| |
|
ויעלה מארבעים ט' אוקי' ושביעית |
|
ויש בהם כסף אוקי' ושביעית |
|
ומן הכסף ישים ב' אוקי' וו' שביעיות |
|
והנה מה שרצינו |
|
ואם רוצה לקשור בד' אוקי' בליט' ויש לו מטבעות שכלם גדולות מד' כגון שיש לו ליט' מה' אוקי' ורביע כסף וליט' מו' וחצי וליט' מז' וג' רביעיות וליט' מח' וחצי ורוצה להתיך מכלם בשוה וצריך לערב נחשת |
|
| |
|
ככה תדענו שתהפוך הענין ותקח חשבון הנחשת אשר במטבעו וחשבון נחשת המטבע אשר הוא רוצה לקשור |
|
וכך הוא החשבון ליט' בג' וחצי נחושת וליט' בד' ורביע נחשת וליט' בה' וחצי נחשת וליט' בו' וג' רביעיות נחשת וליט' מנחושת שהיא י"ב אוקי' ורוצה לקשור מכל זה ד' אוקי' כסף בח' מנחשת |
|
| |
|
כן תעשה חבר נחשת הד' מטבעו יעלה כ' אוקי' |
|
וכפול ליט' הנחשת ד' פעם יעלה מ"ח |
|
חסר המחובר מהכפול ישאר כ"ח ושמרם |
|
ואחר כן חסר הד' מן הי' ישאר ד' |
|
וערכם מהנשאר השמור שביעיות וככה יקח מכל אחד ואחד מהמטבעו' |
|
| |
|
ויעלה לו מארבעתם ו' אוקי' וו' שביעיות |
|
יש בהם מנחשת ב' אוקי' וו' שביעיות |
|
ומן הנחשת ישים ה' אוקי' ושביעית |
|
ויצא מה שרצינו |
| If one produce [a coin] with four [types of coins] and he has some that are less valuable and some that are more valuable, he should sum the less valuable [coins] and multiply one of the most valuable or sum the most valuable [coins] and multiply one of the [less] valuable, then he should proceed according to the rule. | ואם הוא קושר בד' ויש בידו פחותות ויתרות [66]יחבר הפחותות ויכפול אחת מהיתרות או יחבר היתרות ויכפול אחת מהיתרות ויעשה כמשפט |
| You should apply the following rule: multiply according to the number of the coins you mix. | וכלל זה יהא בידך שתכפול הכפול לפי חשבון המטבעות שחברת |
| When you sum the most valuable [coins], subtract the product from the sum, then subtract the one that is less valuable [coin] from the remainder. | ובחברך היתרות תגרע הכפול מהמחובר ותגרע היחידי שהוא פוחת מן הנקשר |
| Vice versa when you sum up the less valuable [coins]: subtract the sum from the product, then subtract the remainder from the one that is the most valuable [coin]. | והפך הדבר בחברך הפחותות שתחסר המחובר מהכפול ותחסר הנקשר מן היחידי היתר |
| From these examples you can deduce for all [kinds of] coins and all human affairs. | ומאלו הדמיונות תוכל להוציא לכל המטבעות ולכל עסקי בני אדם בין רב למע' למעט |
| Instruct a wise man, and he will become wiser [Proverbs 9, 9]. | ותן לחכם ויחכם עוד[note 5] |
Chapter Seven: Conversion of One to the Other |
השער השביעי השבת זה לזה |
|---|---|
The example:
|
והדמיון שאמ' אברכז כי מעלות נטיית הגלגל אשר לשמש אחד עשר חלק משמונים ושלשה בכל הגלגל |
|
ותלמי אמ' כי מעלות הנטייה ארבעים ושבע מעלות ממעלות הגלגל שהם ש"ס ועוד שנים וארבעים חלק ראשונים |
| We wish to know if [their estimates] are equal or different, so do like this: | ונרצה לדעת אם הם שוים או שונים ככה תעשה |
|
ערוך י"א שהוא חשבון אברכז הקטן על מעלות הגלגל שהוא חשבון תלמי הגדול והעולה שהוא ג' אלפים ותשע מאות וששים חלק על פ"ג שהוא חשבון הגדול של אברכז יצא בחלוק מ"ז כדברי תלמי ונשארו נ"ט שלא יתחלקו ערכנום על ששים והעולה שהוא ג' אלפים וחמש מאות וארבעים חלק על פ"ג יצא בחלוק שנים וארבעים חלק כדברי תלמי ונתברר ששני החשבונים שוים |
|
| |
|
והפך הדבר ערכנו מ"ז שהם מעלות תלמי על פ"ג והעולה שהוא ג' אלפים ותשע מאות ואחד וערכנו מ"ב על פ"ג והעולה שהוא ג' אלפים וד' מאות ושמונים וששה חלקנום על ש"ס שהוא החשבון הגדול של תלמי ויצא בחלוק י"א שהוא החשבון הקטן של אברכז והנה שני החשבונים שוים |
Simple fractions to simple fractions |
|
| If you have fractions of the geometricians and you wish to convert them to other fractions | ואם יהיו בידך שברי חכמי המדות ותרצה להשיבם לשברים אחרים |
|
כגון שלשה רובעים כמה שתותים הם |
|
דע מאיזה מספר הוא השתות והוא מששה |
|
קח שלשה רביעיותיו והם ארבעה וחצי וככה שתותים הם |
|
ותדענו שתערוך שלשה על ששה והעולה תחלק על ארבעה והיוצא הם שתותים |
|
ותדענו שתערוך ארבעה על ששה יעלה כ"ד והוא המורה |
|
קח ג' רביעיותיו והם י"ח |
|
חלקם על ששית המורה יצא בחלוק ד' וחצי וככה שתותים הם |
|
ואם תרצה לידע ששה שביעיות כמה תשיעיות הם |
|
דע מאיזה מספר הוא התשיעית והוא מט' |
|
קח ששה שביעיותיו והם שבעה וה' שביעיות תשיעית |
|
או ערוך הששה על תשעה והעולה חלק על שבעה |
|
או ערוך השבעה על תשעה והעולה שהם ס"ג הוא המורה |
|
קח ששה שביעיותיו שהם נ"ד וחלקם על תשיעית המורה שהם ז' יצא שבעה וה' שביעיות |
|
אם כן מצאנו ששה שביעיות הם שבעה תשיעיות וה' שביעיות תשיעית |
|
או דע מאיזה מספר הוא השביעית משבעה |
|
קח ששת שביעיותיו והם ששה |
|
ערכם על תשעה והעולה שהם נ"ד חלק על שבעה יצא לחשבון אחד |
|
ואם תרצה להשיב שבעה שמיניות וחצי שמינית לעשיריות |
|
דע מאיזה מספר הוא העשירית מעשרה |
|
קח שבעה שמיניותיו וחצי שמיניתו יעלה תשעה עשרות ושלשה שמיניות עשירית |
|
ובכל הדרכים האחרים תוכל להוציאו |
| You can solve in these ways if you want to convert sixths into quarters, or ninths into quarters, whether you convert the fractions into smaller or into larger fractions; the [method] is the same. | ועל אלו הדרכים כמו כן תוכל להוציא אם תרצה להשיב ששיות לרביעיות או תשיעיות לרביעיות בין שתשיב השברים לפחותים מהם בין שתשיבם לגדולים מהם הדבר שוה |
Simple fractions to sexagesimal fractions |
|
| If you have simple fractions [= fractions of the geometricians] and you wish to convert them into fractions of the astrologers that are sexagesimal. | ואם יהיו בידיך שברי חכמי המדות ותרצה להשיבם לשברי חכמי המזלות שהם ששים |
| If the fractions you have are a half, a third, a quarter, a fifth, a sixth, or a tenth, it is easy to convert them into sexagesimal [fractions], because they are [divisors of sixty]. | ואם השברים שבידך הם חצי או שליש או רביע או חומש או ששית ועשירית דבר קל להשיב אל ששים מפני שיתחלקו עליו |
| But, if you have a seventh, an eighth, or a ninth, which [are not divisors of] sixty, and you need to know how you can convert them into sexagesimal [fractions]: | אך אם יש בידך שביעית ושמינית ותשיעית לא יתחלקו עליהם ששים ואתה צריך לדעת איך תוכל להשיבם אל ששים |
|
כגון שהיו בידך ב' שביעיות ותרצה להשיבם לחשבון ששים |
|
ככה תעשה ערוך מספר השביעיות על ששים והעולה שהוא ק"כ חלק על שבעה יצא י"ז וישאר אחד |
|
וערוך הנשאר על ששים וחלק על ז' יצא ח' והם שניים ישאר ד' |
|
וערכם על ששים וחלק על ז' יצא ל"ד והם שלשים |
|
| |
| ועל זה הדרך תוכל לדקדק אותו לרביעים או לחמישיים עד אין קץ | |
|
|
או אם תרצה ערוך מספר השביעיים על ח' ל"ד י"ז שהם שביעית ששים יעלה י"ז ח' ל"ד כדרך הראשון |
| ובאלו הדרכים תוציא כמו כן לשמיניות ולתשיעיות | |
|
|
ואם יהיו בידך חלקים משבעים ותרצה להשיבם לחשבון ששים ערוך החלקים על ששים וחלק העולה על שבעים |
|
ואם ישאר שלא יתחלק ערוך על ס' וחלק על ע' יהיו שניים מחשבון ששים |
|
|
או ערוך החלקים שיש בידך על ח' ראשונים ל"ד שניים י"ז שלישיים שהם שביעית ששים וחלק העולה על ששים והיוצא הוא המבוקש |
|
או חסר שביעית החשבון והנשאר הם חלקים מששים |
Chapter Eight: Roots |
השער השמיני שרש זה וזה |
|---|---|
| Extracting roots - integers or fractions - is difficult | השרשים הם קשים בין בשלמים בין בשברים |
| Most of them do not have a known root in the units, the tens, or the consequent ranks, therefore we extract them by approximation. | ורבם אין להם שורש ידוע באחדים ובעשרות ובכל המערכות הבאות אחריהן ונלקחום בדרך קרובה אל האמת |
Integers |
|
| Shortcuts for finding the root of a perfect square | |
| We start to explain the [extraction of roots of] integers that have a known root and give introductions and checks, as eyes for their seekers. | ונחל לפרש השלמים שיש להם שורש ידוע ונתן מפתחות ומאזנים להיות למבקשיהם עינים |
| The roots [of perfect squares] are found in two ways: | והשרשים ימצאו על שני דרכים |
|
האחד על דרך מספר האחדים |
|
והמרובעים הנמצאים בשלמים במערכתם הם שלשה והם א'ד'ט' ושרשם א'ב'ג' |
|
והדרך השני על דרך מספר העשרות |
|
והמרובעים הנמצאים במערכתם ששה והם י"ו כ"ה ל"ו מ"ט ס"ד פ"א ושרשם ד'ה'ו' ז'ח'ט' |
| These are the foundations of all the roots. | ואלה הם מוסדי כל השרשים |
| From here on: every rank that is not even [i.e. every odd rank] takes after the units and every even [rank] takes after the tens. | ומכאן ואילך כל מעלה שאינה זוג היא כאחדים ומה שהיה זוג היא כעשרות |
|
כי המאות כמו האחדים כי הם כמערכת השלישית כמספר נפרד |
|
והאלפים כמו העשרות |
|
ועשרות אלפים כאחדים |
|
ומאות אלפים כעשרות |
|
ואלפי אלפים כאחדים |
|
ועשרות אלפי אלפים כעשרות |
|
ומאות אלפי אלפים כאחדים |
|
ואלף אלפים כעשרות |
|
ככה עד אין קץ ובין כזוג ובין כמה שאינה זוג |
| The [units] that are [roots] of the first rank become ten[s] [as roots] of the third rank, which is analogous to it; hundreds [as roots] of the fifth rank; and thousands [as roots] of the seventh rank. | האחד שהוא במערכת הראשונה ישוב עשרה במעלה השלישית שהיא דומה לה ובמעלה חמישית ממנה מאות ובשביעית ממנה אלפים |
|
ששרש אחד הוא אחד ישוב המעלה השלישית עשרה והוא שורש מאה ששניהם דומים לאחד |
|
ושרש ארבעה שנים על כן שרש ד' מאות עשרים |
|
ועל זה הדרך שרש תשע מאות ל' |
|
והמעלה הרביעית שהיא זוג דומה לשנית |
|
והנה אלף ושש מאות דומה לי"ו על כן שרשו ארבעים |
| כי שב האחד עשרה כמערכת השלישית מן השנית | |
|
|
והנה עשרת אלפים יהיה שרשו מאה כי הוא במעלה החמישית מן הראשונה |
|
|
ויהיה אלף אלפים שהיא המעלה השביעית שרשו אלף |
|
והנה ארבעים אלף כדמות ארבעה |
|
ומאה וששים אלף כדמות ששה עשר ועל זה הדרך לכל המרובעים |
find out if a number is a perfect square
|
וכאשר יהיה בידך חשבון ותרצה לדעת אם יש לו שרש שלם שקלו במאזני תשעה והוא שתחשב האחדים והעשרות והמאות וכל החשבונות כאלו הם אחדים והוציאם ט'ט' |
|
ואם נשאר א' או ד' או ט' או ז' יתכן להיותו מרובע |
|
ואם נשאר ב' או ג' או ה' או ח' דע שאיננו מרובע והנה דרך אחד |
|
ודרך שנית שתסתכל בחשבון אם יש שם ממספרי האחדים שהם במערכת הראשונה |
|
ואם מצאת שם א' או ד' או ה' או ו' או טיתין ט' יתכן להיות מרובע |
|
ודרך שלישית שתדע כי אם יהיה בחשבון א' ראוי להיות בשרש א' או ט' |
| You can know which [digit] will be [the first digit of] the root of the number through the closest to the similar to the square of the units [= the square of a product of a power of ten by a unit, which is the closest to the given number]:
|
ותוכל לדעת איזה יהיה בשרש החשבון לאיזה חשבון הוא קרוב אל הדומה למרובע האחדים |
|
ואם יהיה המרובע הדומה קודם החשבון ויש ביניהם כפל שרש המרובע הוסף על השרש הדומה אחד |
|
ואם היה פחות גרע משרש הדומה אחד |
|
ואם יהיה בשרש ט' |
|
כמו מרבע ת'מ'א' |
|
שקלנוהו במאזני ט' ויצא כלו ט'ט' והנה לאות שיתכן להיותו מרבע |
|
והדומה הקרוב הוא ד' מאות ושרשו כ' |
|
ובעבור שהחשבון גדול ממנו כפל השרש נוסיף בשרש אחד והנה השרש כ"א |
|
| |
|
ובחינתו שתסיר מרבע התוספת מן החשבון ישאר ת"מ |
|
ולו היה החשבון פחות ממרובע הדומה |
|
כגון שס"א |
|
ובעבור שהוא פחות תחסר אחד משורש מרובע הדומה הקרוב שהוא כ' וישאר בשרש ט' והוא י"ט |
|
| |
|
והבחינה להסיר מהחשבון מרובע החסרון וישאר ש"ס ונכפול השרש הדומה והוא מ' וככה מרחק החשבון מן הדומה |
|
ואם במרובע ד' יהיה בשרש ב' שהוא שני לאחד או ח' שהוא שני ליט' |
|
ואם חשבון הדומה לפניו כמו מרובע ת'פ'ד' יהיה בשרש ב' והשרש כ"ב |
|
|
וכשתחסר מרובע התוספת מהחשבון ישאר ת"פ והנה המרחק מן הדומה פ' וככה ראוי להיות כפל הכפל מהשרש הדומה |
|
ואם חשבון הדומה אחריו כמו מרובע ש'כ'ד' שהדומה הקרוב כ' ובעבור שהחשבון פחות חסר ממנו ב' יהיה בשרש ח' והשרש י"ח |
|
|
וכשתסיר מרובע החסרון מהחשבון ישאר ש"כ ונכפול פעמים שרש הדומה וככה המרחק |
|
ואם יש במרובע ט' יהיה בשרש ג' או ז' שהוא רחוק מעשרה ג' לאחורי' |
|
ואם הדומה הקרוב הוא לפניו כגון מרובע ת'ק'כ'ט' שהדומה הקרוב הוא ד' מאות יהיה בשרש ג' והשרש כ"ג |
| וכשתסיר מרובע התוספת מן החשבון ישאר ת'ק' והנה המרחק ק"כ ונכפול שרש הדומה והוא מ' וראוי להיות המרחק ג' פעמים כפל השרש וכן הוא | |
|
| |
|
ואם הדומה הקרוב לאחריו כגון מרובע ת'ש'כ'ט' שהדומה הקרוב הוא ל' ובעבור שהוא פחות חסר ממנו ג' יהיה בשרש ז' והשרש כ"ז |
|
|
ואם תסיר מרובע החסרון מהחשבון ישאר ת'ש'כ' והנה המרחק ק'פ' ונכפול שרש הדומה והוא מ' וראוי להיות המרחק ג' פעמים כפל השרש וככה הוא |
|
ואם במרובע ה' יהיה בשרש ה' בין לפניו בין לאחריו |
|
ואם הוא לפניו כגון אלף ור'כ'ה' שהדומה הוא ל' |
|
וכשתסיר מרובע ה' ישאר אלף ור' ותוספת המרחק הוא ש' |
|
ונכפול השורש הדומה והוא ס' וראוי להיות המרחק ה' פעם ס' וככה הוא |
|
| |
|
ואם הדומה הקרוב לאחריו כגון ת'ר'כ'ה' שהדומה הוא ד' |
|
|
וכשתסיר מרובע ה' ישאר ת'ר' וחסרון המרחק |
|
וכן ראוי להיות כי כל מרובע ה' בין שני מרובעים הדומים |
| כי מספר ת'ר'כ'ה' כאשר נסיר מרובעו שהוא כ"ה נשאר ת"ר והוא בין ד' מאות שהוא הדומה ובין ט' מאות שהוא הדומה האחד לפנים והאחד לאחור מן השרש | |
|
| |
Approximations |
|
| [It seems that the beginning of the discussion concerning this issue is missing though there is no indication for that in the manuscript] | |
|
|
|
|
|
שאינו מתוקן ישאר אחד וב' ששיות וחצי ששית והוא השרש המתוקן |
|
|
ואם תרצה לתקן אותו תקנהו פעם אחרת וערוך חצי הששית שיצא בחלוק על עצמו והעולה חלק על כפל השרש המתוקן והיוצא בחלוק חסר מן השורש המתוקן והנשאר הוא השרש המדוקדק |
| the "corrected root" can be corrected again and again, but yields only an approximation. It allows one to get as close to the answer as one wishes but never to reach the exact answer itself | ואם תרצה תוכל לדקדק אותו כרצונך אך לא תוכל להגיע אל האמת כי לעולם יהיה בדרך קרובה |
|
ואם החשבון שתרצה להוציא שרשו יותר קרוב מהמרובע אשר לאחריו מן המרבע אשר לפניו |
|
כמו שלשה שהוא קרוב אל המרובע ארבעה אשר לאחריו יותר ממרובע אחד אשר לפניו |
|
לעולם תקח מן הקרוב וככה תעשה |
|
קח השרש אשר לארבעה והוא שנים ואחר כן קח המרחק אשר בין חשבונך ובין המרובע שלקחת שרשו וחלקהו על כפל השרש |
|
ותקנהו פעם אחרת שתקח מרובע מה שיצא בחלוק וחלקהו על כפל השרש והיוצא תחסר מהשרש המתוקן |
| You can correct it and make it more accurate as you wish. | ותוכל לתקנו ולדקדק אותו כרצונך |
|
[if the given number is larger than the closest square |
ואם החשבון שתרצה להוציא שרשו הוא מן הזוגות והדומה לו במעלה הרביעית אלף והמרובע הקרוב אליו ת'ת'ק'ס'א' ושרשו ל"א ונשאר ל"ט |
| חלקנום על כפל השרש שהוא ס"ב ויצא חצי אחד ונשארו ח' | |
| עשינו מהם שביעיות והנם נ"ו חלקנום על כפל השרש ונתן לו בדרך קרובה אחד והנה שרש אלף ל"א וחצי ושביעית | |
|
| |
|
|
ועשיריתם בדרך קרובה אל האמת שלשה ושביעית שביעית |
|
ותדענו שתקח שורש המרובע הקרוב אליו והוא ט' ושרשו ג' חלק הנשאר שהוא אחד על כפל השרש יצא ששית |
|
ותקן אותו שתערוך מה שיצא בחלוק על עצמו יעלה ששית הששית חסרהו מן השרש שאינו מתוקן ישאר ג' והם ששיות הששית |
| תקנהו פעם אחרת שתערוך ששית הששית על עצמו ותחסרהו מן השרש המתוקן והנשאר הוא מדוקדק | |
| ואם המרובע הבא לאחריו הוא יותר קרוב מהמרובע שעבר שהוא לפניו תעשה כאשר הראיתיך והדבר שוה בין בנפרדים בין בזוגות | |
Fractions |
|
simple fractions |
|
|
ואם תרצה להוציא שרש ו' שמיניות ושמינית שמינית |
|
דע מאיזה חשבון יצא שמיניתו שמינית השמינית והוא ס"ד ושרשו ח' וקח מן ס"ד ו' שמיניות ושמינית שמיניתו והוא מ"ט |
|
| |
|
ותדענו שתקח כפל כפלו והוא ג' וחצי שמינית ושרשו א' וג' רביעיות |
|
| |
|
|
ואם תרצה להוציא שרש שנים וחצי |
| דע כי החצי יצא משנים ואין לשנים שרש ידוע ונעשה מהכל חציים ויעלו ה' נערכם על שנים ויעלו י' ושרשם ג' וששית בדרך קרובה ונחלק זה השרש על שנים יצא אחד וחצי וחצי ששית והוא דרך קרובה | |
|
| |
|
|
ואם תרצה להוציא שרש אחד וג' חומשים |
| דע כי החומש יצא מה' נעשה מכלם חמישיות יהיו ח' נערכם על ה' יעלו מ' ושרשם בדרך קרובה ו' שליש, חלקם על ה' יצא אחד וחומש ושליש חומש | |
|
| |
|
|
ותדענו שתערוך המ' בק' וקח שרש העולה וחלקהו על העולה מערך ה' על שרש ק' והיוצא הוא השרש |
| ועל אלו הדרכים נהגו חכמי המדות להוציא השרשים בשלמים ובנשברים ובשברי השברים עד אין קץ | |
sexagesimal fractions |
|
| The astrologers extract it by degrees, minutes, seconds, thirds | וחכמי המזלות מוציאים אותו למעלות לראשונים ולשניים ולשלישיים ומדקדקים עד עשיריים ועד כמה שירצו |
| וסוף הכל לא יגיעו אל האמת רק בדרך קרובה | |
| extracting roots of degrees is the same as extracting roots of integers | וכאשר אתה מוציא שרש מספר מעלות, אתה מוציאו בדרך הוצאת שרשי המספר השלם ויהיה השרש מעלות כמוהו |
| two kinds of sexagesimal fractions: | וכאשר תוציא שרש מספר שברים יש לך לדעת כי השברים נחלקים לשני מינין: |
|
יש מהם שיש להם שרש ידוע ומפורסם |
|
כגון השניים והרביעים ששורש השניים הם ראשונים ושרש רביעיים הם שניים |
|
מפני כי כאשר תערוך ראשונים על ראשונים יעלו שניים |
|
וכאשר תערוך שניים על שניים יעלו רביעים |
|
ויש מהם שאין להם שרש ידוע ומפורסם |
|
כגון הראשונים והשלישיים והחמישיים וכל הדומה להם |
|
כי אין אתה מוצא שברים שתהיה עריכתם על עצמם מוציאה אל השברים האלה |
|
ומיכן היה הדרך בהוצאת שרשי השברים אם יהיו השברים שתרצה להוציא שרשם מן המין ששרשם ידוע ומפורסם אתה מוצא שרשם בדרך הוצאת שרשי מספרי השלמים |
| אך שאתה קורא שם השרש ממין השברים אשר עריכתו על עצמו מוציאך אל שם השברים שאתה רוצה להוציא שרשם | |
|
כגון שהיית רוצה להוציא ט' שניים |
|
ואתה יודע כי שרש ט' במספר השלם הוא ג' שלמים ובכאן אתה אומר כי השרש ו' ראשונים |
| ועל זה הדרך לכל השברים אשר שרשם ידוע ומפורסם | |
|
ואם היו השברים שאתה מוציא שרשיהם מן השברים הסתומים והנעלמים שאין שרשיהם מפורסמים |
|
אתה משיב השברים ההם אל השברים הקרובים אשר פחותים מהם ששרשיהם ידועים ויהיה שרש המספר ההוא מן המין אשר עריכתו על עצמו מוציאך אל המין אשר החזרת חשבונך אליו |
|
כאלו רצית להוציא שרש ט"ו שלישיים והם מן השברים הסתומים |
|
ואתה מחזיר אותם אל רביעיים אשר הוא הקרובים אליהם והם מהידועים וכשנערוך ט"ו על ששים יעלו תת"ק רביעיים ושרשם הוא שלשים והם שניים |
| ועל זה הדרך לכל השברים שאין שרשם מפורסם | |
|
ולעולם אם יהיה במרובע שניים יהיה בשרש ראשונים |
|
ואם רביעיים יהיה בשרש שניים |
|
והכלל כי בשרש יהיה מספר חצי השברים |
sexagesimal approximations of integers |
|
| Two sexagesimal approximation methods | והנה לך שני דרכים על דרך חכמי המזלות להוציא השרשים בדרך קרובה מדוקדקים היטב |
First approximation method |
|
|
| |
|
הדרך האחד שתוציא שרש המרובע שעבר הקרוב אליו והנשאר שהוא המרחק שיש בין חשבונך ובין המרובע תערך אל ששים יקראו ראשונים והעולה תחלק על כפל השרש |
| והשמר שישאר שתוכל לחסר מרובע מה שיצא בחלוק | |
| וככה עד שתגיע אל השלישים והוא מדוקדק | |
|
והדמיון רצינו להוציא שרש שנים |
|
והמרובע הקרוב שעבר הוא אחד ושרשו אחד ונשאר אחד ונשאר א' נשיבהו ראשונים והוא ששים |
|
והנה יוסיפו כ"ה שניים |
|
ובעבור זה לא נתננו לו כי אם כ"ד ונשארו י"ב ומרובע כ"ד הוא ט' ראשונים ל"ו שניים |
|
| |
|
השיבונו ב' ראשונים כ"ד שניים לשלישיים בעבור שנוכל לחלק על כפל השרש שהם ק'ס'ח' ראשונים יצא בחלוק נ"א שניים ונשארו לנו ע"ב שלישיים |
|
| |
|
|
ועל זה הדרך תדקדקהו תמצא השרש א'כ'ד' נ"א י'ח' רביעיים והוא מדוקדק |
| ואם תערוך על זה המספר שרש חשבון שתרצה שיהיה העולה שרש כפל החשבון | |
|
|
ואם תשיב האחד לס' ראשונים ותחבר עמהם הכ"ד ראשונים יהיו פ"ד ותחשבם שהם שלמים ותחשב כמו כן השניים ראשונים והשלישיים שניים והרביעיים שלישיים אז תמצא שרש שבעת אלפים ומאתים כי שלשת אלפים ושש מאות הוא מרובע ששים והנו חשוב כאחד והמספר הנזכר והוא כפלו |
|
| |
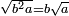
|
ולעולם אם ידענו שרש מספר ונרצה לדעת שרש מספר שהוא כפל כפלו נכפול השרש וככה הוא |
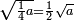
|
והפך הדבר אם ידענו שרש מספר ידוע ונרצה לדעת כמה שרש מספר שהוא רביעיתו נקח חצי השרש וככה הוא |
Second approximation method |
|
|
והדרך האחרת |
|
|
|
חלק המרחק מהמרובע הקרוב על כפל השרש ותן לו הכל והיוצא תחבר עם השרש הקרוב אשר לפניו וככה השרש שאיננו מתוקן |
|
אך אם היה המרובע הבא אשר לאחריו יותר קרוב |
|
תחלק המרחק על שרש המרובע הבא והיוצא תחסר מהשורש מן המרובע הבא והנשאר הוא השרש שאיננו מתוקן |
|
ואחר כן קח מה שעלה בחלוק על איזה דרך שיהיה וערוך אותו על עצמו והעולה תחלק על כפל השרש שאיננו מתוקן |
| ועל זה הדרך תקנהו עד שתגיע לשלישיים והוא מדוקדק קרוב אל האמת | |
checking whether the given number is larger than the closest square 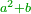 or less than the closest square or less than the closest square 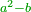
|
ובהוציאך השרש תוכל לדעת ולהכיר אם לקחת חשבון קרוב אל המרובע שעבר או אל הבא |
|
כי כאשר תחלק המרחק ממרובע הראשון על כפל השרש אם עלה יותר משלשים אז תדע כי הוא קרוב אל המרובע הבא |
|
ואם עלה שלשים או פחות דע כי הוא קרוב אל המרובע שעבר |
|
והדמיון רצינו להוציא שרש שניים |
|
יהיה המרחק אחד והוא ס' ראשונים חלקנום על כפל השרש שהוא שנים |
|
|
|
|
רצינו לתקנו ערכנו ל' על עצמו וחלקנום על ס' יצא ט"ו |
|
| |
|
|
|
|
נתקן אותו פעם שנית ונערוך ה' ראשונים שיצאו בחלוק על עצמם עלו כ"ה והם שניים |
|
השיבונו אותם שלישיים עלו אלף וחמש מאות |
|
וחלקנום על כפל השרש המתוקן והוא ק"ע ראשונים יצא בחלוק ח' והם שניים ונשארו ק"מ |
|
השיבונום לרביעים עלו ח' אלפים וארבע מאות |
|
חלקנום על ק"ע ויצא מ"ט ונשארו עוד לחלק אך אין צריך להטריח יותר |
|
| |
|
|
והנה יש לנו לחסר מהשרש המתוקן ח' שניים מ"ט שלישיים וישאר א'כ"ד כ"א י"א והוא מדוקדק |
|
|
| ואם תרצה להוציא שרשו שתקח דמיונו במעלה השלישית והוא במאות והנכון במעלה החמישית ואם תקחנו במעלה השביעית יהיה יותר נכון | |
|
וכאשר תוציא שרש שנים מן המעלה החמישית תוציא שרש עשרים אלף שהוא הדומה כמשפט |
|
ולדעת שרש שנים חלק הכל על מאה והיוצא הוא שרש שנים והוא אחד שלם כ"ד ראשונים נ"א שניים י' שלישיים ז' רביעיים מ"ח חמישיים |
|
ואם ידעת שרש שנים תערכנו על מאה והעולה הוא שרש כ' אלף |
|
ואם הוצאת שרש שנים מן המעלה השלישית שהיא המאות הוציא שרש מאתים שהוא הדומה |
|
והיוצא חלק על עשרה ונמצא שרש שנים |
|
ואם ידעת שרש שנים תערכנו על מאה והעולה הוא שרש כ' אלף |
|
ואם הוצאת שרש שנים מן עשרה והעולה הוא שורש מאתים |
| ואם תרצה להוציא שרש עשרה תוציאנו בכל הדרכים כמשפטם תמצאנו ג' שלמים כ"ו ראשונים מ"ד שניים י"ב שלישיים | |
Shortcuts |
|
| For every number that we know its root, if we multiply it by ten and we wish to know how much is the root of the product, multiply the root of the number by the root of ten and the result is the required.
|
וכל מספר שנדע שרשו אם נערכנו על עשרה ונרצה לדעת כמה שרש המחובר מהמערכות תערוך השרש של המספר על שרש עשרה והעולה הוא המבוקש |
there are tables of roots from 1 to 2½ and a range up to 6¼: 
|
והנה יש לוחות עשויות מאחד שלם עד שנים וחצי ויעלה המרובע עד ששה שלמים ורביע |
| guidelines for finding roots using these tables: | |
|
ואם היו לך חלקים פחותים מאחד ערכם על ארבעה וקח בלוח שרש העולה ומה שיהיה קח חציו |
|
ואם כפלנוהו כל ככה ולא עלה עד אחד ערכהו על שמונה וקח רביעית השרש |
|
ואם היה חשבונך מאחד ועד ששה ורביע ותרצה להוציא השרש, בקש מספר חשבונך |
|
ואם לא תמצאנו קח השרש בלוח שהוא כנגד החשבון הקרוב הנמצא לפניו או לאחריו וקח הקרוב אל חשבונך בין לפניו בין לאחריו וערוך המרחק הנמצא בין החשבון ובין חשבונך על ששים וחלק העולה על המרחק הנמצא בין שני המרובעים וההוה תוסיפנו על השרש הנמצא אם היה החשבון בלוחות שלקחת פחות מחשבונך ואם יותר תגרענו אז תמצא המבוקש |
|
ואם היה המספר יותר מששה ורביעית עד חמשה ועשרים שלמים קח שרש רביעיתו וההוה בשרש כפלהו תמצא השרש |
|
ואם היה יותר מכ"ה עד מאה כפלהו ארבעה פעמים וקח שרש הדומה וההוה קח חציו וככה שרש המבוקש |
| והנה המאות דומות לאחדים | |
|
ואם היה לך מספר יותר ממאה ערכהו על ששה וחלק העולה על עשרה וההוה קח שרשו וקח לכל מאה אחד במרובע וכאשר תדע השרש תערכנו על עשרה אז תמצא השרש המבוקש |
|
ואם היה חשבונך באלפים חשוב כי הוא בעשרות והוצא דמיונו והיוצא ערכנו על עשרה אז תמצא רצונך |
|
ואם החשבון בעשרות אלפים הוציא הדומה וההוה תערכנו על מאה |
|
ואם במאות אלפים הוצא הדומה בעשרות וההוה תערכנו על מאה |
| ועל זה הדרך תוכל להוציא שרש כל חשבון שתרצה | |
|
ואלף אלפים דומה לאחדים וההוה בשרש ערכנו על אלף |
| ומשם והלאה תעשה על זה הדרך | |
תהלה לאל עולם
Notes
Apparatus
Appendix I: Glossary of Terms
Appendix II: Bibliography
Anonymous Textbook
Manuscripts:
- Budapest, Magyar tudomanyos akademia, MS Kaufmann A 507/2 (IMHM: f 15161), ff. 20-43 (15th-16th century)
- Genève, Bibliothèque de Genève, MS héb. 10/1 (IMHM: f 2320), ff. 2r-38r (14th-15th century)
- Vatican, Biblioteca Apostolica, MS ebr. 171/18 (IMHM: f 8630), f. 104r, line 3 – f. 105v, line 19 (Canea, 1493)
The transcript is based mainly on manuscript Genève 10
Bibliography:
- Aradi, Naomi. 2013. An Unknown Medieval Hebrew Anonymous Treatise on Arithmetic, Aleph 13.2, pp. 235-309.
- Lévy, Tony. 2002. A Newly Discovered Partial Hebrew Version of al-Khwārizmı̄’s Algebra, Aleph 2, pp. 225–34.


![\scriptstyle{\color{OliveGreen}{n=\left[\frac{1}{2}\sdot\left(n-1\right)\right]+\left[\frac{1}{2}\sdot\left(n+1\right)\right]}}](/mediawiki/images/math/e/c/f/ecf15d858a99040f9ad8e644b76e7a48.png)
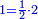

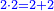

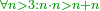
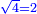
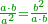
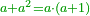
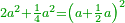

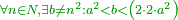
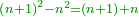
![\scriptstyle{\color{OliveGreen}{\left[\left(n-1\right)\sdot\left(n+1\right)\right]+1=n^2}}](/mediawiki/images/math/1/f/b/1fbc3c3780f1396f2a3d7dff682a2226.png)

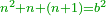
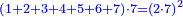
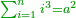
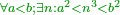
![\scriptstyle{\color{OliveGreen}{a=\left[\left(\frac{1}{2}n\right)-\frac{1}{2}\right]\sdot n}}](/mediawiki/images/math/a/9/a/a9acadf85e2c5569112d90a372248590.png)
![\scriptstyle{\color{OliveGreen}{b=\left[\left(\frac{1}{2}n\right)+\frac{1}{2}\right]\sdot n}}](/mediawiki/images/math/a/d/b/adb0d5616a4ba87fdc754424d4df6844.png)
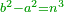
![\scriptstyle{\color{OliveGreen}{\left(n+1\right)^3-n^3=\left(n+1\right)^2+n^2+\left[\left(n+1\right)\sdot n\right]}}](/mediawiki/images/math/7/8/b/78b7139239f29f3d86631815640809f2.png)
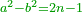
![\scriptstyle{\color{OliveGreen}{\left[\left(a^2-b^2\right)\sdot a\right]+\left[\left(a^2-b^2\right)\sdot b\right]}}](/mediawiki/images/math/3/0/c/30c95e1da47187a6465db586a4250f87.png)
![\scriptstyle{\color{OliveGreen}{a=b+1\longrightarrow\left[\left(a^2-b^2\right)\sdot a\right]+\left[\left(a^2-b^2\right)\sdot b\right]=\left(a^2-b^2\right)^2}}](/mediawiki/images/math/1/b/3/1b33b258ee33352763bbcc76bbdf6a89.png)
![\scriptstyle{\color{OliveGreen}{a=b+2\longrightarrow2\sdot\left[\left[\left(a^2-b^2\right)\sdot a\right]+\left[\left(a^2-b^2\right)\sdot b\right]\right]=\left(a^2-b^2\right)^2}}](/mediawiki/images/math/0/c/a/0ca8b2f020d853c550a4fd704dba85b8.png)
![\scriptstyle{\color{OliveGreen}{a=b+3\longrightarrow3\sdot\left[\left[\left(a^2-b^2\right)\sdot a\right]+\left[\left(a^2-b^2\right)\sdot b\right]\right]=\left(a^2-b^2\right)^2}}](/mediawiki/images/math/8/0/8/808ea48f0d81c8301bbca84aa263e5f9.png)
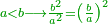

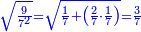


![\scriptstyle{\color{OliveGreen}{a>b\longrightarrow a^2=\left[\left(a-b\right)\sdot a\right]+\left[\left(a-b\right)\sdot b\right]+b^2}}](/mediawiki/images/math/b/f/a/bfa549196c3269dad8f2a3750f64ec25.png)
![\scriptstyle{\color{OliveGreen}{a<b\longrightarrow a^2=b^2-\left[\left(b-a\right)\sdot a\right]-\left[\left(b-a\right)\sdot b\right]}}](/mediawiki/images/math/6/9/7/697653ecf969cbfa9e3f21cf93250796.png)
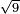
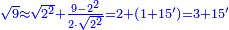
![\scriptstyle{\color{blue}{\frac{2\sdot\left[1^2+\left(2\sdot15^\prime\right)+\left[2\sdot\left(15^\prime\right)^2\right]\right]}{2\sdot\left[2\sdot\left(3+15^\prime\right)\right]}=\frac{2\sdot\left[1+30^\prime+\left(7^\prime+30^{\prime\prime}\right)\right]}{13}=\frac{2\sdot\left(1+37^\prime+30^{\prime\prime}\right)}{13}=\frac{2\sdot\left(97^\prime+30^{\prime\prime}\right)}{13}=\frac{195^\prime}{13}=15^\prime}}](/mediawiki/images/math/1/0/0/10033b77bba0b955971292c46fc87f44.png)
![\scriptstyle{\color{blue}{\sqrt{9}=\left(3+15^\prime\right)-\frac{2\sdot\left[1^2+\left(2\sdot15^\prime\right)+\left[2\sdot\left(15^\prime\right)^2\right]\right]}{2\sdot\left[2\sdot\left(3+15^\prime\right)\right]}=\left(3+15^\prime\right)-15^\prime=3}}](/mediawiki/images/math/6/7/9/67903f0c471f4b35f08226743216c2ca.png)


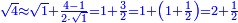
![\scriptstyle{\color{blue}{\sqrt{4}=\left(2+\frac{1}{2}\right)-\frac{1^2+\left(2\sdot\frac{1}{2}\right)+\left[2\sdot\left(\frac{1}{2}\right)^2\right]}{2\sdot\left(2+\frac{1}{2}\right)}=\left(2+\frac{1}{2}\right)-\frac{2+\frac{1}{2}}{2\sdot\left(2+\frac{1}{2}\right)}=\left(2+\frac{1}{2}\right)-\frac{1}{2}=2}}](/mediawiki/images/math/6/b/8/6b8ad8f5d55d03e88ce26fcf7a247423.png)
![\scriptstyle{\color{OliveGreen}{a^2-\left[a+\left(a-1\right)\right]=b^2}}](/mediawiki/images/math/6/3/2/6329c80cd4e2d19fd96fa02f79b37aec.png)
![\scriptstyle{\color{OliveGreen}{a^2+\left[a+\left(a+1\right)\right]=c^2}}](/mediawiki/images/math/2/9/7/2970beb5f398434f29290ae0322a3feb.png)
![\scriptstyle{\color{OliveGreen}{a^2=10a-\left[\left(10-a\right)\sdot a\right]}}](/mediawiki/images/math/6/0/5/605517108bb09392281d796df0be0449.png)
![\scriptstyle{\color{blue}{9^2=\left(9\sdot10\right)-\left[\left(10-9\right)\sdot9\right]=90-\left(1\sdot9\right)=81}}](/mediawiki/images/math/e/4/1/e41fa1d31a6cf398eef9c61aa78e26cf.png)
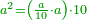
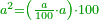
![\scriptstyle{\color{blue}{75^2=\left(\frac{75}{100}\sdot75\right)\sdot100=\left(\frac{3}{4}\sdot75\right)\sdot100=\left[75-\left(18+\frac{3}{4}\right)\right]\sdot100=\left(56+\frac{1}{4}\right)\sdot100=5625}}](/mediawiki/images/math/1/5/3/153a2cb31735bfe4e95a8391abe4d365.png)

![\scriptstyle{\color{blue}{16:10=1+\frac{3}{5}=\frac{\left(6+\frac{1}{4}\right)+\left[\frac{3}{5}\sdot\left(6+\frac{1}{4}\right)\right]}{6+\frac{1}{4}}=10:\left(6+\frac{1}{4}\right)}}](/mediawiki/images/math/7/a/3/7a3bd5130ce987474d6469769499dfbb.png)
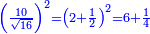
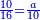
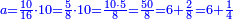
![\scriptstyle{\color{blue}{\frac{16}{10}\sdot\left(6+\frac{1}{4}\right)=\left(1+\frac{3}{5}\right)\sdot\left(16+\frac{1}{4}\right)=\left(16+\frac{1}{4}\right)+\left[\frac{3}{5}\sdot\left(6+\frac{1}{4}\right)\right]=10}}](/mediawiki/images/math/f/e/d/fedf7320425943539905a4f6efb65aa1.png)
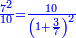
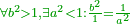
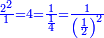
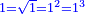
 ] and no subtraction [
] and no subtraction [ ].
].
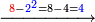
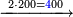

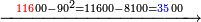
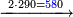
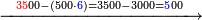

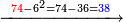

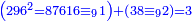
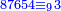


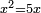
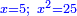
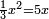
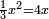
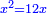
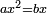
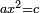
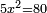
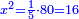
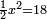
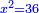
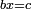


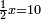
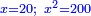
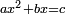
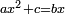
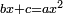
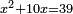


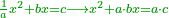
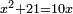

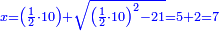
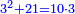
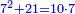

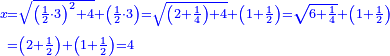
![\scriptstyle{\color{OliveGreen}{\sum_{i=1}^{2n-1} i=\left(2n-1\right)\sdot\left[\left[\frac{1}{2}\sdot \left(2n-1\right)\right]+\frac{1}{2}\right]}}](/mediawiki/images/math/5/6/b/56bb680020be3e2fc0e2790841bd4562.png)
![\scriptstyle{\color{blue}{\sum_{i=1}^{11} i=n\sdot\left[\left(\frac{1}{2}\sdot11\right)+\frac{1}{2}\right]=11\sdot6=66}}](/mediawiki/images/math/0/6/f/06f970bc7137159a2ab14aa652cfbfa6.png)
![\scriptstyle{\color{OliveGreen}{\sum_{i=1}^{2n-1} i=\left[\left(2n-1\right)+1\right]\sdot\left[\frac{1}{2}\sdot\left(2n-1\right)\right]}}](/mediawiki/images/math/d/6/b/d6ba7a681352b67fe283573e33b7f63d.png)
![\scriptstyle{\color{OliveGreen}{\sum_{i=1}^{2n} i=\left[2n\sdot\left(\frac{1}{2}\sdot2n\right)\right]+\left(\frac{1}{2}\sdot2n\right)}}](/mediawiki/images/math/3/d/8/3d8aae7e2ed76f3d5ec4c12b2f4f1f5c.png)
![\scriptstyle{\color{blue}{\sum_{i=1}^{10} i=\left[10\sdot\left(\frac{1}{2}\sdot10\right)\right]+\left(\frac{1}{2}\sdot10\right)=\left(10\sdot5\right)+5=55}}](/mediawiki/images/math/b/b/6/bb68f216b91f76482347d0d5dfe0b742.png)

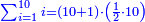


![\scriptstyle{\color{OliveGreen}{\sum_{k=1}^{n} 2k=\left[\left(\frac{1}{2}\sdot2n\right)+\left(\frac{1}{2}\sdot2\right)\right]\sdot\left(\frac{1}{2}\sdot2n\right)}}](/mediawiki/images/math/2/8/d/28d76309c66cfd0f833d5ca1c298f741.png)
![\scriptstyle{\color{blue}{\sum_{i=1}^{10} 2i=\left[\left(\frac{1}{2}\sdot20\right)+\left(\frac{1}{2}\sdot2\right)\right]\sdot\left(\frac{1}{2}\sdot20\right)=\left[10+\left(\frac{1}{2}\sdot2\right)\right]\sdot10=11\sdot10}}](/mediawiki/images/math/a/0/a/a0aef8c7a8ea1e69a3e9ff5f91feb418.png)
![\scriptstyle{\color{OliveGreen}{\sum_{i=1}^{n} 2i=\left[\left(\frac{1}{2}\sdot n\right)+\frac{1}{2}\right]\sdot2n}}](/mediawiki/images/math/3/9/b/39b705c06ca37b802afeb48889b6ded8.png)
![\scriptstyle{\color{blue}{\sum_{i=1}^{10} 2i=\left[\left(\frac{1}{2}\sdot10\right)+\frac{1}{2}\right]\sdot20=\left(5+\frac{1}{2}\right)\sdot20}}](/mediawiki/images/math/1/e/7/1e7a8c3e9658295cde7f79d3fb27ef4f.png)
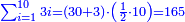
![\scriptstyle{\color{OliveGreen}{\sum_{i=1}^{n} 3i=\left[\left(\frac{1}{2}\sdot3n\right)+\left(\frac{1}{2}\sdot3\right)\right]\sdot n}}](/mediawiki/images/math/1/0/1/10162f6c170194f0bae48da061d06c26.png)
![\scriptstyle{\color{blue}{\sum_{i=1}^{10} 3i=\left[\left(\frac{1}{2}\sdot30\right)+\left(\frac{1}{2}\sdot3\right)\right]\sdot\left(\frac{1}{3}\sdot30\right)=\left[15+\left(\frac{1}{2}\sdot3\right)\right]\sdot10=\left(16+\frac{1}{2}\right)\sdot10}}](/mediawiki/images/math/8/7/6/876200dd8bf8a7613490421edf150e14.png)
![\scriptstyle{\color{OliveGreen}{\sum_{i=1}^{n} 4i=\left[\left(\frac{1}{2}\sdot\frac{1}{4}\sdot an\right)+\frac{1}{2}\right]\sdot 4n}}](/mediawiki/images/math/c/5/e/c5ec303844b7e9b62f1e7c81b5f0b32c.png)
![\scriptstyle{\color{OliveGreen}{\sum_{i=1}^{n} 5i=\left[\left(\frac{1}{2}\sdot\frac{1}{5}\sdot 5n\right)+\frac{1}{2}\right]\sdot 5n}}](/mediawiki/images/math/b/a/f/baf5de85497a8a52fd9baaf877bdc75e.png)
![\scriptstyle{\color{OliveGreen}{\sum_{i=1}^{n} ai=\left[\left(\frac{1}{2}\sdot\frac{1}{a}\sdot an\right)+\frac{1}{2}\right]\sdot an}}](/mediawiki/images/math/4/1/6/416d0cd5481e534c789cc5ad432c5d33.png)
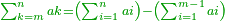
![\scriptstyle{\color{blue}{\sum_{i=10}^{19} i=\left(\sum_{i=1}^{19} i\right)-\left[\left[\frac{1}{2}\sdot\left(10-1\right)\right]\sdot10\right]=190-\left[\left[\frac{1}{2}\sdot\left(10-1\right)\right]\sdot10\right]}}](/mediawiki/images/math/a/1/5/a155b42978ccbefa5bb6188b676c9234.png)


![\scriptstyle{\color{blue}{\sum_{i=5}^{10} 2i=110-\left[\left[\frac{1}{2}\sdot\left(10-2\right)\right]\sdot\left(\frac{1}{2}\sdot10\right)\right]}}](/mediawiki/images/math/9/8/a/98a419691d798e578b4a89b09cc4dfab.png)
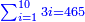
![\scriptstyle{\color{blue}{\sum_{i=4}^{10} 3i=465-\left[\left[\frac{1}{2}\sdot\left(12-3\right)\right]\sdot\left(\frac{1}{3}\sdot12\right)\right]}}](/mediawiki/images/math/6/9/b/69bb994c6027afd7ff801965e6f09024.png)
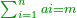
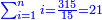
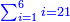
 and the sum is 315.
and the sum is 315.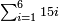
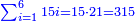
![\scriptstyle{\color{OliveGreen}{\sum_{i=1}^{2n+1} 2^{i-1}=\left[2\sdot\left(2^{\frac{\left(2n+1\right)-1}{2}}\right)^2\right]-1=\left[2\sdot2^{2n}\right]-1}}](/mediawiki/images/math/6/c/9/6c9437ea6fc92be61b5a01c7a0e95f42.png)
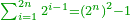
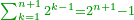
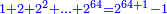
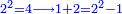
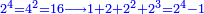

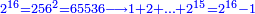
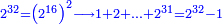

![\scriptstyle{\color{OliveGreen}{\sum_{k=1}^{n} k^2=\left[\left(n+1\right)\sdot\left(\frac{1}{2}\sdot n\right)\right]\sdot\left[\left(\frac{2}{3}\sdot n\right)+\frac{1}{3}\right]}}](/mediawiki/images/math/8/c/a/8ca1d983fcff11845ed7123640c7c7cf.png)
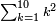
![\scriptstyle{\color{blue}{\sum_{k=1}^{10} k^2=\left[\left(10+1\right)\sdot\left(\frac{1}{2}\sdot10\right)\right]\sdot\left[\left(\frac{2}{3}\sdot10\right)+\frac{1}{3}\right]=55\sdot7}}](/mediawiki/images/math/e/4/6/e462dcd61583e0b569458db8623e1619.png)
![\scriptstyle{\color{OliveGreen}{\sum_{k=m}^{n} k^2=\sum_{k=1}^{n} k^2-\left[\left[\left[\left(m-1\right)\sdot\frac{1}{2}\right]\sdot m\right]\sdot\left[\left[\frac{2}{3}\sdot\left(m-1\right)\right]+\frac{1}{3}\right]\right]}}](/mediawiki/images/math/7/b/7/7b70c413dcf9fd97d3582bafa9d3a611.png)
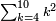
![\scriptstyle{\color{blue}{\sum_{k=4}^{10} k^2=\sum_{k=1}^{10} k^2-\left[\left[\left[\left(4-1\right)\sdot\frac{1}{2}\right]\sdot4\right]\sdot\left[\left(\frac{2}{3}\sdot3\right)+\frac{1}{3}\right]\right]}}](/mediawiki/images/math/3/0/8/308b968aa002a8d3bacc0210087a52ab.png)
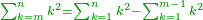
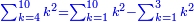
![\scriptstyle{\color{blue}{\sum_{k=5}^{10} k^2=\sum_{k=1}^{10} k^2-\left[\left[\left[\left(5-1\right)\sdot\frac{1}{2}\right]\sdot5\right]\sdot\left[\left(\frac{2}{3}\sdot4\right)+\frac{1}{3}\right]\right]}}](/mediawiki/images/math/5/7/8/578c2c53ee5ee93be3e312bdda94bd4b.png)
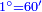
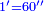
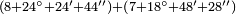
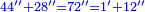
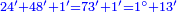



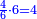
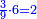
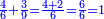
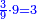
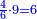
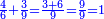

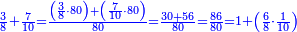
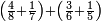
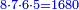




![{\color{blue}{\begin{align}\scriptstyle\left(\frac{4}{8}+\frac{1}{7}\right)+\left(\frac{3}{6}+\frac{1}{5}\right)&\scriptstyle=\frac{\left[\left(\frac{4}{8}\sdot1680\right)+\left(\frac{1}{7}\sdot1680\right)\right]+\left[\left(\frac{3}{6}\sdot1680\right)+\left(\frac{1}{5}\sdot1680\right)\right]}{1680}\\&\scriptstyle=\frac{1080+1176}{1680}=\frac{2256}{1680}=1+\frac{2}{7}+\left(\frac{1}{4}\sdot\frac{1}{7}\right)+\left(\frac{1}{2}\sdot\frac{1}{4}\sdot\frac{1}{7}\right)+\left(\frac{1}{10}\sdot\frac{1}{4}\sdot\frac{1}{7}\right)\\\end{align}}}](/mediawiki/images/math/9/e/0/9e0906e615770823e29a177630299753.png)
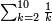
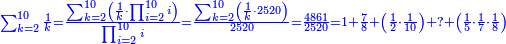

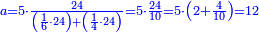
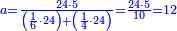
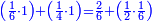
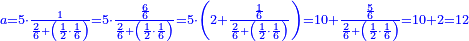

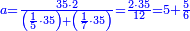
![\scriptstyle{\color{blue}{a=2\sdot\frac{35}{\left(\frac{1}{5}\sdot35\right)+\left(\frac{1}{7}\sdot35\right)}=2\sdot\frac{35}{12}=2\sdot\left[3-\left(\frac{1}{2}\sdot\frac{1}{6}\right)\right]=5+\frac{5}{6}}}](/mediawiki/images/math/f/6/8/f6820c46198e5ece587b1f47daa1711f.png)
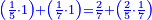
![\scriptstyle{\color{blue}{a=2\sdot\frac{1}{\frac{2}{7}+\left(\frac{2}{5}\sdot\frac{1}{7}\right)}=2\sdot\left[2+\frac{\frac{2}{7}+\left(\frac{1}{5}\sdot\frac{1}{7}\right)}{\frac{2}{7}+\left(\frac{2}{5}\sdot\frac{1}{7}\right)}\right]=4+\frac{\frac{4}{7}+\left(\frac{2}{5}\sdot\frac{1}{7}\right)}{\frac{2}{7}+\left(\frac{2}{5}\sdot\frac{1}{7}\right)}=4+\left[1+\frac{\frac{10}{5}\sdot\frac{1}{7}}{\frac{2}{7}+\left(\frac{2}{5}\sdot\frac{1}{7}\right)}\right]=5+\frac{10}{12}}}](/mediawiki/images/math/a/c/b/acb8488409ce44a0500121d10d911df0.png)
![\scriptstyle\left[\left(\frac{1}{3}\sdot\frac{1}{4}\sdot\frac{1}{5}\right)\sdot a\right]+\left[\left(\frac{1}{7}\sdot\frac{1}{8}\sdot\frac{1}{9}\right)\sdot a\right]=2](/mediawiki/images/math/7/9/4/794cfeb38a33298248c8beb2bbcaaf4d.png)
![{\color{blue}{\begin{align}\scriptstyle a&\scriptstyle=2\sdot\frac{2520}{\left[\left(\frac{1}{3}\sdot\frac{1}{4}\sdot\frac{1}{5}\right)\sdot2520\right]+\left[\left(\frac{1}{7}\sdot\frac{1}{8}\sdot\frac{1}{9}\right)\sdot2520\right]}=2\sdot\frac{2520}{42+5}=2\sdot\frac{2520}{47}\\&\scriptstyle=2\sdot\left(53+\frac{29}{47}\right)=106+\frac{58}{47}=106+1+\frac{11}{47}=107+\frac{11}{47}\\\end{align}}}](/mediawiki/images/math/3/5/0/350b83ba141d1a45e93d53d160a416b9.png)
![\scriptstyle{\color{blue}{a=\frac{2\sdot2520}{\left[\left(\frac{1}{3}\sdot\frac{1}{4}\sdot\frac{1}{5}\right)\sdot2520\right]+\left[\left(\frac{1}{7}\sdot\frac{1}{8}\sdot\frac{1}{9}\right)\sdot2520\right]}=\frac{2\sdot2520}{47}=107+\frac{11}{47}}}](/mediawiki/images/math/3/d/a/3da4de584f88ba936061416abf22589b.png)
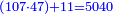
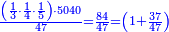
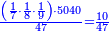
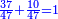
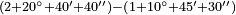
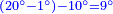
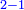
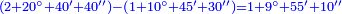
![\scriptstyle10-\left[\left(\frac{1}{6}\sdot10\right)+\left(\frac{1}{4}\sdot10\right)\right]](/mediawiki/images/math/a/1/d/a1d97ff50871513400365ebcd487aa8a.png)
![\scriptstyle{\color{blue}{12-\left[\left(\frac{1}{6}\sdot12\right)+\left(\frac{1}{4}\sdot12\right)\right]=7}}](/mediawiki/images/math/3/3/d/33dfcec01093bb32ff598181b158bec3.png)
![\scriptstyle{\color{blue}{10-\left[\left(\frac{1}{6}\sdot10\right)+\left(\frac{1}{4}\sdot10\right)\right]=\frac{7}{12}\sdot10=5+\frac{5}{6}}}](/mediawiki/images/math/d/1/c/d1c612b2ceb20e7224afa166cbae0b72.png)
![\scriptstyle{\color{blue}{10-\left[\left(\frac{1}{6}\sdot10\right)+\left(\frac{1}{4}\sdot10\right)\right]=\frac{10}{12}\sdot7=\frac{5}{6}\sdot7=5+\frac{5}{6}}}](/mediawiki/images/math/0/4/4/044287a70b44b8ce604ae271f631ad0a.png)
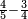
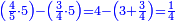


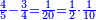
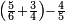
![\scriptstyle{\color{blue}{\left[\left(\frac{5}{6}\sdot30\right)+\left(\frac{3}{4}\sdot30\right)\right]-\left(\frac{4}{5}\sdot30\right)=\left(47+\frac{1}{2}\right)-24=23+\frac{1}{2}}}](/mediawiki/images/math/2/9/c/29c8ab403d3dd1af213d159271381551.png)
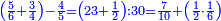
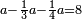


![\scriptstyle{\color{OliveGreen}{a=\frac{n\sdot m}{\left(n\sdot m\right)-\left[\left[\frac{1}{n}\sdot\left(n\sdot m\right)\right]+\left[\frac{1}{m}\sdot\left(n\sdot m\right)\right]\right]}\sdot b}}](/mediawiki/images/math/d/5/a/d5af5089a39514224807c0286cdbbcce.png)
![\scriptstyle{\color{OliveGreen}{a=\frac{b}{\left(n\sdot m\right)-\left[\left[\frac{1}{n}\sdot\left(n\sdot m\right)\right]+\left[\frac{1}{m}\sdot\left(n\sdot m\right)\right]\right]}\sdot\left(n\sdot m\right)}}](/mediawiki/images/math/e/c/7/ec7c26c4065e4cb6a216e65f85134659.png)
![\scriptstyle\left(a-\frac{1}{5}a\right)-\left[\frac{1}{7}\sdot\left(a-\frac{1}{5}a\right)\right]=24](/mediawiki/images/math/3/7/4/374076552ffc3b65b582bafe02e38a00.png)
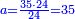
![\scriptstyle{\color{OliveGreen}{\left[a-\left(\frac{1}{n}\sdot a\right)\right]-\left[\frac{1}{m}\sdot\left[a-\left(\frac{1}{n}\sdot a\right)\right]\right]=b}}](/mediawiki/images/math/5/2/1/521bc9ccb2f87c9a4835c93b82a68977.png)
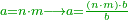
![\scriptstyle{\color{blue}{5\sdot6=30\longrightarrow\left[30-\left(\frac{1}{5}\sdot30\right)\right]-\left[\frac{1}{6}\sdot\left[30-\left(\frac{1}{5}\sdot30\right)\right]\right]=20}}](/mediawiki/images/math/a/8/b/a8b4fb85ba2061c2ee6fd40301c0cb64.png)
![\scriptstyle{\color{OliveGreen}{\left(a-\frac{1}{5}a\right)-\left[\frac{1}{7}\sdot\left(a-\frac{1}{5}a\right)\right]=17+\frac{1}{7}}}](/mediawiki/images/math/f/7/0/f706b47807e8bb8aad8176e20d20442a.png)
![\scriptstyle{\color{blue}{5\sdot7\ne25\longrightarrow\left[25-\left(\frac{1}{5}\sdot25\right)\right]-\left[\frac{1}{7}\sdot\left[25-\left(\frac{1}{5}\sdot25\right)\right]\right]=17+\frac{1}{7}}}](/mediawiki/images/math/9/4/e/94ee8615f27ff9c0e073190ccc9aa7b8.png)
![\scriptstyle{\color{blue}{\left(\frac{1}{5}\sdot35\right)+\left[\frac{1}{7}\sdot\left[35-\left(\frac{1}{5}\sdot35\right)\right]\right]=7+\left[\frac{1}{7}\sdot\left(35-7\right)\right]=7+\left(\frac{1}{7}\sdot28\right)=7+4=11}}](/mediawiki/images/math/b/e/7/be7af84c599a9702a27a9cdea4852dd5.png)
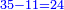

![\scriptstyle{\color{blue}{\left(17+\frac{1}{7}\right)\sdot\left[\frac{1}{4}+\left(\frac{5}{6}\sdot\frac{1}{4}\right)\right]=7+\frac{6}{7}}}](/mediawiki/images/math/f/b/7/fb74968c1148d7314c945145d0608788.png)

![\scriptstyle{\color{OliveGreen}{a=b+\left(\frac{1}{n-1}\sdot b\right)+\left[\frac{1}{m-1}\sdot\left[b+\left(\frac{1}{n-1}\sdot b\right)\right]\right]}}](/mediawiki/images/math/0/f/2/0f2bddd324df91c36cffacf202099513.png)
![\scriptstyle{\color{blue}{a=\left(17+\frac{1}{7}\right)+\left[\frac{1}{6}\sdot\left(17+\frac{1}{7}\right)\right]+\frac{1}{4}\sdot\left[17+\left(\frac{1}{7}\right)+\left[\frac{1}{6}\sdot\left(17+\frac{1}{7}\right)\right]\right]}}](/mediawiki/images/math/b/d/e/bdece7a97d81b3c13872ad944dfb533f.png)
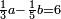
![\scriptstyle{\color{blue}{\begin{cases}\scriptstyle a=\left[\left(15\sdot\frac{1}{5}\right)+6\right]\sdot3=\left(3+6\right)\sdot3=9\sdot3=27\\\scriptstyle \frac{1}{5}b=3\longrightarrow b=15\end{cases}}}](/mediawiki/images/math/3/a/b/3ab9475039c685a7c96f52c9e3661694.png)
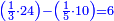
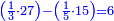
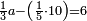
![\scriptstyle{\color{blue}{a=\left[6+\left(\frac{1}{5}\sdot10\right)\right]\sdot3}}](/mediawiki/images/math/9/f/b/9fb3e3e07b1461f5218f62f52845d28d.png)
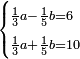


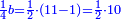
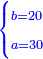
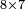
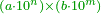
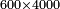

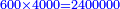
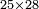

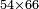
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle54\times66&\scriptstyle=\left[\left(\frac{50}{100}\sdot66\right)\sdot100\right]+\left(4\sdot66\right)=\left[\left(\frac{1}{2}\sdot66\right)\sdot100\right]+264\\&\scriptstyle=\left(33\sdot100\right)+264=3300+264=3564\\\end{align}}}](/mediawiki/images/math/d/2/d/d2dcff5ce76e67e6b7bd557d89eafbb5.png)
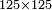
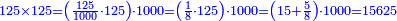
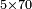
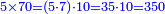

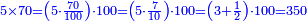
![\scriptstyle{\color{blue}{5\times300=\left(\frac{5}{100}\sdot300\right)\sdot100=\left[\left(\frac{1}{2}\sdot\frac{1}{10}\right)\sdot300\right]\sdot100=15\sdot100}}](/mediawiki/images/math/1/4/6/14612faaf59a164181a05ac6636a133f.png)
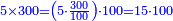
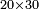
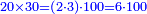
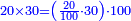

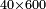
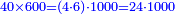

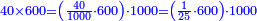

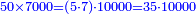


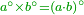
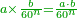
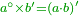

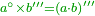
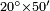

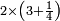

 .
.
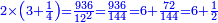
![\scriptstyle{\color{blue}{2\times\left(3+\frac{1}{4}\right)=\frac{2\sdot\left[\left(3\sdot4\right)+1\right]}{4}=\frac{2\sdot\left(12+1\right)}{4}=\frac{2\sdot13}{4}=\frac{26}{4}=6+\frac{1}{2}}}](/mediawiki/images/math/9/e/b/9ebb902113adb9c9b6516f8d3a5d9422.png)
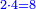
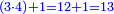 .
.

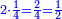
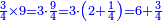

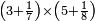
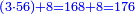
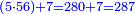



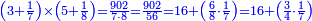
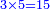
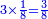
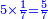
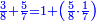
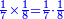
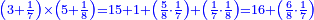
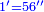
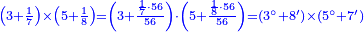
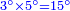
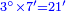
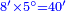
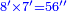
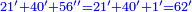
![\scriptstyle{\color{blue}{\left(3+\frac{1}{7}\right)\times\left(5+\frac{1}{8}\right)=15^\circ+62^\prime=15^\circ+1^\circ+6^\prime=16^\circ+6^\prime=\left[16+\left(\frac{3}{4}\sdot\frac{1}{7}\right)\right]^\circ}}](/mediawiki/images/math/a/b/8/ab85e5551c1c68da951d5d947c330f97.png)
![\scriptstyle\left(3+\frac{1}{4}\right)\times\left[5+\left(\frac{1}{6}\sdot\frac{1}{7}\right)\right]](/mediawiki/images/math/a/e/2/ae2eb1d97e60b3daefe628a8a4333262.png)

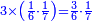

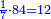
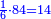
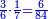

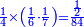
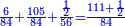
![{\color{blue}{\begin{align}\scriptstyle\left(3+\frac{1}{4}\right)\times\left[5+\left(\frac{1}{6}\sdot\frac{1}{7}\right)\right]&\scriptstyle=15+\frac{111+\frac{1}{2}}{84}=15+\frac{84+27+\frac{1}{2}}{84}=15+1+\frac{27+\frac{1}{2}}{84}\\&\scriptstyle=16+\frac{21+6+\frac{1}{2}}{84}=16+\frac{1}{4}+\frac{6+\frac{1}{2}}{84}\\&\scriptstyle=16+\frac{1}{4}+\left(\frac{2}{7}\sdot\frac{1}{4}\right)+\left(\frac{1}{6}\sdot\frac{1}{7}\sdot\frac{1}{4}\right)\\\end{align}}}](/mediawiki/images/math/6/7/0/67063b710aa1ec41ea429525fe874aea.png)


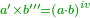
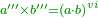
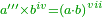

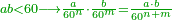
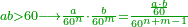
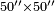
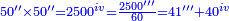
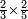
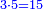


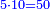

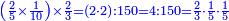
![\scriptstyle\left[\frac{3}{4}\sdot\left(3+\frac{1}{3}\right)\right]\times\left(\frac{6}{7}\sdot5\right)](/mediawiki/images/math/1/a/c/1ac4f88a6d78fe1ca6efd685e4d7383f.png)
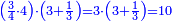
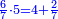
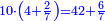
![\scriptstyle{\color{blue}{\left[\frac{3}{4}\sdot\left(3+\frac{1}{3}\right)\right]\times\left(\frac{6}{7}\sdot5\right)=\frac{42+\frac{6}{7}}{4}=10+\frac{5}{7}}}](/mediawiki/images/math/f/8/9/f8955275d28af1f3362cd09d8774bd51.png)
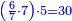
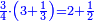
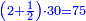
![\scriptstyle{\color{blue}{\left[\frac{3}{4}\sdot\left(3+\frac{1}{3}\right)\right]\times\left(\frac{6}{7}\sdot5\right)=
\frac{75}{7}=10+\frac{5}{7}}}](/mediawiki/images/math/4/2/f/42feda1496f4369722717e3f35bdaba7.png)
![\scriptstyle{\color{blue}{\left[\frac{3}{4}\sdot\left(3+\frac{1}{3}\right)\right]\times\left(\frac{6}{7}\sdot5\right)=\left(2+\frac{1}{2}\right)\sdot\left(4+\frac{2}{7}\right)=10+\frac{5}{7}}}](/mediawiki/images/math/e/9/5/e95370870e15985c0bf40ea4eb7e0f20.png)
![\scriptstyle\left[\frac{7}{8}\sdot\left(5+\frac{1}{3}\right)\right]\times\left[\frac{3}{5}\sdot\left(6+\frac{1}{4}\right)\right]](/mediawiki/images/math/3/c/4/3c44a996a735d16f077b6f5636f1fd0a.png)


![\scriptstyle{\color{blue}{\left[\frac{7}{8}\sdot\left(5+\frac{1}{3}\right)\right]\times\left[\frac{3}{5}\sdot\left(6+\frac{1}{4}\right)\right]=\frac{37+\frac{1}{3}}{8}\sdot\frac{18+\frac{3}{4}}{5}=\frac{139+\frac{4}{4}}{8}=17+\frac{1}{2}}}](/mediawiki/images/math/1/4/2/14226c70721156a36c475d7ed7ea74a5.png)
![\scriptstyle{\color{blue}{\left[\frac{7}{8}\sdot\left(5+\frac{1}{3}\right)\right]\times\left[\frac{3}{5}\sdot\left(6+\frac{1}{4}\right)\right]=\frac{37+\frac{1}{3}}{8}\sdot\frac{18+\frac{3}{4}}{5}=\frac{\left(4+\frac{2}{3}\right)\sdot\left(18+\frac{3}{4}\right)}{5}=\frac{87+\frac{1}{2}}{5}=17+\frac{1}{2}}}](/mediawiki/images/math/2/3/9/23948e19af1ed84f590521c31540bb01.png)
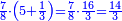

![\scriptstyle{\color{blue}{\left[\frac{7}{8}\sdot\left(5+\frac{1}{3}\right)\right]\times\left[\frac{3}{5}\sdot\left(6+\frac{1}{4}\right)\right]=\frac{14}{3}\sdot\frac{15}{4}=\frac{14\sdot\left(3+\frac{3}{4}\right)}{3}=17+\frac{1}{2}}}](/mediawiki/images/math/4/9/e/49e74c31c3372c8d3ebc3970f1c8b37f.png)
![\scriptstyle{\color{blue}{\left[\frac{7}{8}\sdot\left(5+\frac{1}{3}\right)\right]\times\left[\frac{3}{5}\sdot\left(6+\frac{1}{4}\right)\right]=\frac{14}{3}\sdot\frac{15}{4}=\frac{\left(4+\frac{2}{3}\right)\sdot15}{4}=\frac{70}{4}=17+\frac{1}{2}}}](/mediawiki/images/math/9/8/a/98a6dd3ddabf531dc87d83441bfb8660.png)
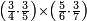

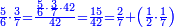
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle\left(\frac{3}{4}\sdot\frac{3}{5}\right)\times\left(\frac{5}{6}\sdot\frac{3}{7}\right)&\scriptstyle=\left[\frac{2}{5}+\left(\frac{1}{4}\sdot\frac{1}{5}\right)\right]\sdot\left[\frac{2}{7}+\left(\frac{1}{2}\sdot\frac{1}{7}\right)\right]=\frac{140\sdot\left[\frac{2}{5}+\left(\frac{1}{4}\sdot\frac{1}{5}\right)\right]\sdot\left[\frac{2}{7}+\left(\frac{1}{2}\sdot\frac{1}{7}\right)\right]}{140}\\&\scriptstyle=\frac{22+\frac{1}{2}}{140}=\frac{1}{7}+\left(\frac{1}{8}\sdot\frac{1}{7}\right)\\\end{align}}}](/mediawiki/images/math/7/3/1/731b480409a79c84beb1d2839dddade1.png)
![\scriptstyle{\color{blue}{\left(\frac{3}{4}\sdot\frac{3}{5}\right)\times\left(\frac{5}{6}\sdot\frac{3}{7}\right)=\frac{9}{20}\sdot\left[\frac{2}{7}+\left(\frac{1}{2}\sdot\frac{1}{7}\right)\right]=\frac{9\sdot\left[\frac{2}{7}+\left(\frac{1}{2}\sdot\frac{1}{7}\right)\right]}{20}=\frac{3+\frac{1}{7}+\left(\frac{1}{2}\sdot\frac{1}{7}\right)}{20}=\frac{1}{7}+\left(\frac{1}{8}\sdot\frac{1}{7}\right)}}](/mediawiki/images/math/1/1/c/11c0904770226283a50ad33b476ea128.png)

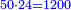
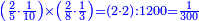
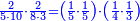
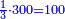

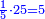
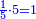
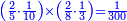
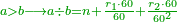
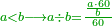

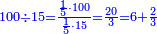

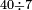
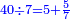

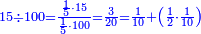
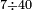
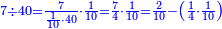
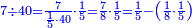
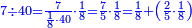
![\scriptstyle{\color{blue}{\left[\frac{2}{10}-\left(\frac{1}{4}\sdot\frac{1}{10}\right)\right]\sdot40=7}}](/mediawiki/images/math/e/3/7/e37b262131999cf0c3e5d10be7365d57.png)
![\scriptstyle{\color{blue}{\left[\frac{1}{5}-\left(\frac{1}{8}\sdot\frac{1}{5}\right)\right]\sdot40=7}}](/mediawiki/images/math/0/2/9/029e15d45923fbee4bbda30e80fa69c8.png)
![\scriptstyle{\color{blue}{\left[\frac{1}{8}+\left(\frac{2}{5}\sdot\frac{1}{8}\right)\right]\sdot40=7}}](/mediawiki/images/math/f/9/f/f9fc4f8fb00d95d78afdbce6f5bab3a2.png)
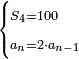

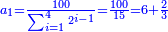
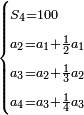
![\scriptstyle{\color{blue}{1+\left(1+\frac{1}{2}\right)+\left[\left(1+\frac{1}{2}\right)+\frac{1}{3}\sdot\left(1+\frac{1}{2}\right)\right]+\left[\left[\left(1+\frac{1}{2}\right)+\frac{1}{3}\sdot\left(1+\frac{1}{2}\right)\right]+\frac{1}{4}\sdot\left[\left(1+\frac{1}{2}\right)+\frac{1}{3}\sdot\left(1+\frac{1}{2}\right)\right]\right]=7}}](/mediawiki/images/math/e/e/3/ee333d0a1e3daaccc1b747ff0a5db333.png)
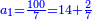


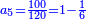
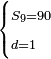
![\scriptstyle{\color{blue}{\sum_{i=1}^{9-1} i=\sum_{i=1}^{8} i=\left[\left(8+1\right)\sdot\left(\frac{1}{2}\sdot8\right)\right]=36}}](/mediawiki/images/math/4/9/0/490595aaecc6da7c8c1107abcdf56489.png)
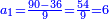
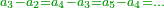 ]
]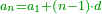 ]
]![\scriptstyle{\color{OliveGreen}{a_1=\frac{S_n-\sum_{i=1}^{n-1} i}{n}=\frac{S_n-\left[n\sdot\left[\frac{1}{2}\sdot\left(n-1\right)\right]\right]}{n}}}](/mediawiki/images/math/7/4/d/74d1638f038ded38066b767ecff46acd.png) ]
]
![\scriptstyle{\color{blue}{a_1=\frac{80-\left[3+\left(3+1\right)+\left(3+1+2\right)+\left(3+1+2+6\right)\right]}{5}=\frac{80-25}{5}=\frac{55}{5}=11}}](/mediawiki/images/math/3/1/1/3117596a6b3e1b03ad8e76096769ff87.png)
![\scriptstyle a_1=\frac{S_3^2-\left[2a_1+\left[a_2\sdot\left(S_3-1\right)\right]+\left(a_3\sdot S_3\right)\right]}{S_3-2}](/mediawiki/images/math/7/4/b/74b406a92903345a162d5a49fefd60be.png)


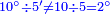

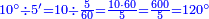


![\scriptstyle20\div\left[\frac{2}{3}\sdot\left(7+\frac{1}{5}\right)\right]](/mediawiki/images/math/2/4/c/24c083330efd4b6463fe3289713dd1a5.png)
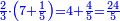
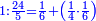
![\scriptstyle{\color{blue}{\scriptstyle20\div\left[\frac{2}{3}\sdot\left(7+\frac{1}{5}\right)\right]=20\sdot\left[\frac{1}{6}+\left(\frac{1}{4}\sdot\frac{1}{6}\right)\right]=4+\frac{1}{6}}}](/mediawiki/images/math/a/d/a/ada019ee97e0df4b80c349cdf8f539a1.png)
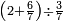
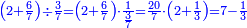
![\scriptstyle\left(2+\frac{1}{2}\right)\div\left[\frac{7}{8}+\left(\frac{1}{2}\sdot\frac{1}{8}\right)\right]](/mediawiki/images/math/d/8/e/d8e83feda1d6610aa137ca89b047e05e.png)
![{\color{blue}{\begin{align}\scriptstyle\left(2+\frac{1}{2}\right)\div\left[\frac{7}{8}+\left(\frac{1}{2}\sdot\frac{1}{8}\right)\right]&\scriptstyle=\frac{20}{8}\sdot\frac{1}{\frac{7}{8}+\left(\frac{1}{2}\sdot\frac{1}{8}\right)}=\frac{20}{8}\sdot\left[1+\left(\frac{1}{3}\sdot\frac{1}{5}\right)\right]=\frac{21}{8}+\left(\frac{1}{3}\sdot\frac{1}{8}\right)\\&\scriptstyle=2+\frac{5}{8}+\left(\frac{1}{3}\sdot\frac{1}{8}\right)=3-\frac{1}{3}\\\end{align}}}](/mediawiki/images/math/a/e/b/aeb22c83f7afacafcd9a79a7e5e607e9.png)
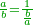
![\scriptstyle a\sdot\left[\frac{2}{5}+\left(\frac{1}{10}\sdot\frac{1}{5}\right)\right]=1](/mediawiki/images/math/3/7/7/3778176acc4bf275d05b23058a9d3c17.png)
![\scriptstyle{\color{blue}{\left[\frac{2}{5}+\left(\frac{1}{10}\sdot\frac{1}{5}\right)\right]\sdot50=21}}](/mediawiki/images/math/5/a/6/5a62aaa9b864834e8b9a434ccd6637d7.png)
![\scriptstyle{\color{blue}{a\sdot\left[\frac{2}{5}+\left(\frac{1}{10}\sdot\frac{1}{5}\right)\right]=1\longrightarrow a=\frac{50}{21}=2+\frac{1}{3}+\left(\frac{1}{7}\sdot\frac{1}{3}\right)}}](/mediawiki/images/math/6/7/1/671108f23ce267b018217d47dc4431ee.png)
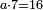
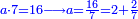
![\scriptstyle\left[\frac{3}{4}\sdot\left(\frac{3}{5}\sdot9\right)\right]\div24](/mediawiki/images/math/f/d/5/fd5da07e5ee7c1d16ab429c699e3a165.png)
![\scriptstyle{\color{blue}{\left[\frac{3}{4}\sdot\left(\frac{3}{5}\sdot9\right)\right]\div24=\left(\frac{3}{4}\sdot\frac{27}{5}\right)\div24=\frac{4+\left(\frac{1}{2}\sdot\frac{1}{10}\right)}{24}}}](/mediawiki/images/math/2/5/b/25bc66f9b17c777073d5d4939e202a09.png)
![\scriptstyle{\color{blue}{\frac{4+\left(\frac{1}{2}\sdot\frac{1}{10}\right)}{24}=\frac{\frac{8\sdot\left[4+\left(\frac{1}{2}\sdot\frac{1}{10}\right)\right]}{24}}{8}=\frac{1}{8}+\left(\frac{1}{3}\sdot\frac{1}{8}\right)+\left(\frac{1}{2}\sdot\frac{1}{10}\sdot\frac{1}{3}\sdot\frac{1}{8}\right)}}](/mediawiki/images/math/2/2/8/228b3484f8b9a8825d222f1daefb5ce6.png)
![\scriptstyle{\color{blue}{\frac{4+\left(\frac{1}{2}\sdot\frac{1}{10}\right)}{24}=\frac{\frac{3\sdot\left[4+\left(\frac{1}{2}\sdot\frac{1}{10}\right)\right]}{24}}{3}=\left(\frac{1}{2}\sdot\frac{1}{3}\right)+\left(\frac{1}{2}\sdot\frac{1}{10}\sdot\frac{1}{3}\sdot\frac{1}{8}\right)}}](/mediawiki/images/math/7/0/f/70fb2be6e47e7834af1a1bd5811ee4fd.png)
![\scriptstyle{\color{blue}{\frac{4+\left(\frac{1}{2}\sdot\frac{1}{10}\right)}{24}=\frac{\frac{4\sdot\left[4+\left(\frac{1}{2}\sdot\frac{1}{10}\right)\right]}{24}}{4}=\left(\frac{2}{3}\sdot\frac{1}{4}\right)+\left(\frac{1}{2}\sdot\frac{1}{10}\sdot\frac{1}{6}\sdot\frac{1}{4}\right)}}](/mediawiki/images/math/f/4/e/f4e230dc5199ceaee1aa5ab8073b1949.png)

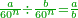
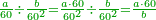
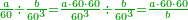
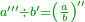
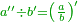
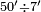
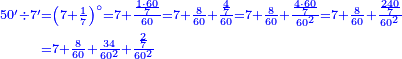
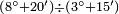
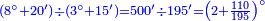
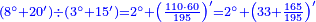
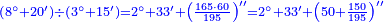


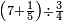
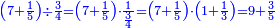
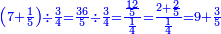
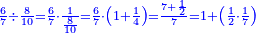
![\scriptstyle{\color{blue}{\frac{6}{7}\div\frac{8}{10}=\frac{\frac{6}{8}}{7}\div\frac{1}{10}=\frac{1-\frac{1}{4}}{7}\div\frac{1}{10}=\left[\frac{1}{7}-\left(\frac{1}{4}\sdot\frac{1}{7}\right)\right]\div\frac{1}{10}=\frac{7}{7}+\left(\frac{1}{2}\sdot\frac{1}{7}\right)}}](/mediawiki/images/math/b/1/7/b17759b34258c56758a694f42702a9d7.png)
![\scriptstyle\left[\frac{6}{7}\sdot\left(5+\frac{1}{4}\right)\right]\div\left[\frac{3}{8}\sdot\left(1+\frac{1}{5}\right)\right]](/mediawiki/images/math/a/c/7/ac790ab4f0c5e266ccc6cb6b836c03b1.png)
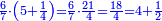
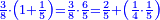
![\scriptstyle{\color{blue}{\left[\frac{2}{5}+\left(\frac{1}{4}\sdot\frac{1}{5}\right)\right]\sdot\left(2+\frac{2}{9}\right)=1}}](/mediawiki/images/math/0/5/9/0594e524801206478f581c1e90e3ea1d.png)
![\scriptstyle{\color{blue}{\frac{1}{4}\sdot\frac{1}{5}=\frac{1}{9}\sdot\left[\frac{2}{5}+\left(\frac{1}{4}\sdot\frac{1}{5}\right)\right]}}](/mediawiki/images/math/7/1/2/712a2bb1daa78bf98f67982635a279e7.png)
![\scriptstyle{\color{blue}{\left[\frac{6}{7}\sdot\left(5+\frac{1}{4}\right)\right]\div\left[\frac{3}{8}\sdot\left(1+\frac{1}{5}\right)\right]=\left(4+\frac{1}{2}\right)\sdot\left(2+\frac{2}{9}\right)=10}}](/mediawiki/images/math/5/c/0/5c0630351a5a57e6b1a4c41e1256d012.png)


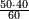
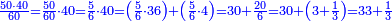
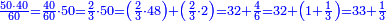
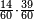
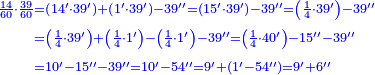
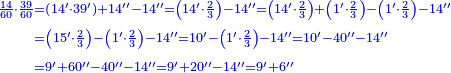

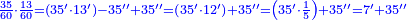
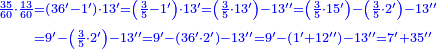
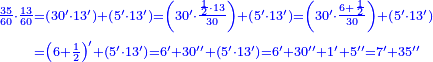

![{\color{blue}{\begin{align}\scriptstyle\frac{46}{60}\sdot\frac{25}{60}&\scriptstyle=46^\prime\sdot\left[\frac{1}{3}+\left(\frac{1}{4}\sdot\frac{1}{3}\right)\right]=46^\prime\sdot\frac{1}{3}\sdot\left(1+\frac{1}{4}\right)=\left(15^\prime+20^{\prime\prime}\right)\sdot\left(1+\frac{1}{4}\right)\\&\scriptstyle=\left(15^\prime+20^{\prime\prime}\right)+\left(15^\prime+20^{\prime\prime}\right)\sdot\frac{1}{4}=15^\prime+20^{\prime\prime}+3^\prime+50^{\prime\prime}=19^\prime+10^{\prime\prime}\\\end{align}}}](/mediawiki/images/math/b/2/4/b242e0b3a88a8979f61e74ae450a26a7.png)
![{\color{blue}{\begin{align}\scriptstyle\frac{46}{60}\sdot\frac{25}{60}&\scriptstyle=\left(46^\prime\sdot24^\prime\right)+\left(46^\prime\sdot1^\prime\right)=46^\prime\sdot\left(\frac{2}{5}+1^\prime\right)=\left[45^\prime\sdot\left(\frac{2}{5}+1^\prime\right)\right]+\left[1^\prime\sdot\left(\frac{2}{5}+1^\prime\right)\right]\\&\scriptstyle=18^\prime+45^{\prime\prime}+\left[1^\prime\sdot\left(24^\prime+1^\prime\right)\right]=18^\prime+45^{\prime\prime}+24^{\prime\prime}+1^{\prime\prime}=19^\prime+10^{\prime\prime}\\\end{align}}}](/mediawiki/images/math/5/f/4/5f41627b7f4ed9e8a2a97d01463117d8.png)


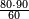
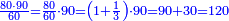
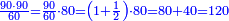
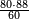
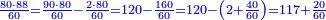
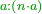
![\scriptstyle{\color{OliveGreen}{a:\left[\left(1+\frac{1}{m}\right)\sdot a\right]}}](/mediawiki/images/math/f/c/4/fc4d1259c62a36e18bfa2717d538557b.png)

![\scriptstyle{\color{OliveGreen}{a:\left[\left(n+\frac{1}{m}\right)\sdot a\right]}}](/mediawiki/images/math/4/9/f/49ffeb341070689e9bd7477550d14286.png)

![\scriptstyle{\color{OliveGreen}{a:\left[\left(1+\frac{r}{m}\right)\sdot a\right]}}](/mediawiki/images/math/8/8/1/881413e48793148bcf7349859fda67c3.png)

![\scriptstyle{\color{OliveGreen}{a:\left[\left(n+\frac{r}{m}\right)\sdot a\right]}}](/mediawiki/images/math/b/b/e/bbe4ec31fcd206b9fcf1bc44b6803a41.png)

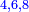
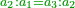
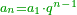
![\scriptstyle{\color{blue}{9:6:4\longrightarrow q=1+\frac{1}{2}\longrightarrow6:4=\left[4\sdot\left(1+\frac{1}{2}\right)\right]:4=\left[6\sdot\left(1+\frac{1}{2}\right)\right]:6=9:6}}](/mediawiki/images/math/7/5/6/756be9b5b3e5a8677075f50931c07469.png)
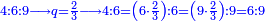

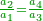

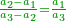
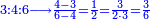
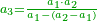
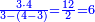
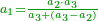
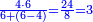
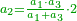
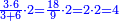
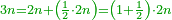


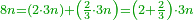
 ], since its one side is equal to its other side, and when you count its side by its multitude the product is the square number.
], since its one side is equal to its other side, and when you count its side by its multitude the product is the square number. ].
]. ], since it has no other side but one, which is not a number.
], since it has no other side but one, which is not a number.
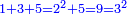




![\scriptstyle{\color{OliveGreen}{x_1=a_3-\left[a_2+\left(a_2-a_1\right)\right]}}{\color{blue}{=9-\left[6+\left(6-4\right)\right]=1}}](/mediawiki/images/math/c/6/d/c6d3dc69d0bc528ebf471016106220a5.png)
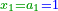
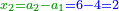
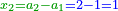
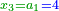
![\scriptstyle{\color{OliveGreen}{x_3=a_3-\left[a_2+\left(a_2-a_1\right)\right]}}{\color{blue}{=4-\left[2+\left(2-1\right)\right]=1}}](/mediawiki/images/math/b/8/b/b8bb8e5a3852eeef74fd7287e34f27f8.png)
![\scriptstyle{\color{OliveGreen}{\sum_{k=1}^n k=n\sdot\left[\left(\frac{1}{2}\sdot n\right)+\frac{1}{2}\right]}}](/mediawiki/images/math/f/a/6/fa6baa34b565d43b5520a33eb7e7b4dc.png)
![\scriptstyle{\color{blue}{6\sdot\left[\left(\frac{1}{2}\sdot6\right)+\frac{1}{2}\right]=21}}](/mediawiki/images/math/a/e/a/aea414d9e1212dae3c7a94b10896734c.png)
![\scriptstyle{\color{blue}{5\sdot\left[\left(\frac{1}{2}\sdot5\right)+\frac{1}{2}\right]=5\sdot3=15}}](/mediawiki/images/math/9/3/b/93b7a01eee210e0c8b3564be3a95258b.png)
![\scriptstyle{\color{blue}{4\sdot\left[\left(\frac{1}{2}\sdot4\right)+\frac{1}{2}\right]=4\sdot\left(2+\frac{1}{2}\right)=10}}](/mediawiki/images/math/8/d/c/8dcb9fc5daf2d06f013534f9070a4a28.png)
![\scriptstyle{\color{blue}{3\sdot\left[\left(\frac{1}{2}\sdot3\right)+\frac{1}{2}\right]=3\sdot2=6}}](/mediawiki/images/math/8/4/9/8498e8411722f69ceec740ca7148af49.png)
![\scriptstyle{\color{blue}{2\sdot\left[\left(\frac{1}{2}\sdot2\right)+\frac{1}{2}\right]=2\sdot\left(1+\frac{1}{2}\right)=3}}](/mediawiki/images/math/6/5/1/6519f9961d55250aa0c0e946d8d00aa1.png)
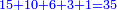



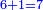
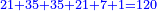

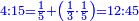

![\scriptstyle{\color{OliveGreen}{\frac{\left[amount\ of\ goods\ offered\ in\right]\ business}{price}=\frac{sold\ \left[amount\ of\ goods\right]}{money\ \left[paid\right]}}}](/mediawiki/images/math/6/a/4/6a4de5708c8e2857cb12749f97b5fd4d.png)



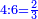
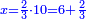
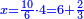
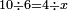
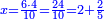
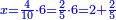
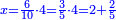

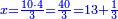
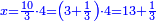
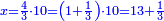
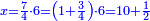
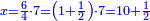

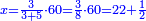


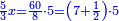
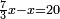
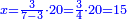

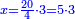
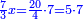
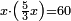
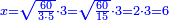


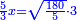
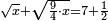

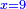
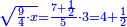
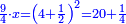
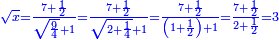
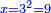
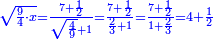

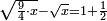
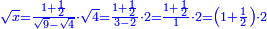

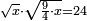
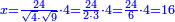
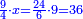
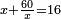
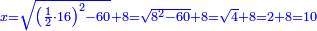
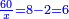
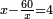
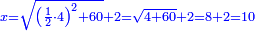
![\scriptstyle3x+\left[7\sdot\left(1-x\right)\right]=6](/mediawiki/images/math/0/3/8/03896ab071ff585de4d04aa27ed3415e.png)
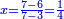
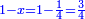

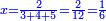
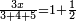
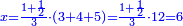
![\scriptstyle\left[\left(3+7\right)\sdot x\right]+\left[12\sdot\left(1-2x\right)\right]=10](/mediawiki/images/math/8/0/2/8025bb98c833778586376b3608eac629.png)
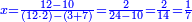

![\scriptstyle\left[\left(2+3+5\right)\sdot x\right]+\left[14\sdot\left(1-3x\right)\right]=12](/mediawiki/images/math/b/f/3/bf35d2d99dd4cbfbb45254630ea49227.png)

![\scriptstyle{\color{blue}{1-3x=1-\left[3\sdot\left(\frac{1}{2}\sdot\frac{1}{8}\right)\right]=\frac{6}{8}+\left(\frac{1}{2}\sdot\frac{1}{8}\right)}}](/mediawiki/images/math/b/e/9/be982293d267e02fe60ed804f20d2e26.png)

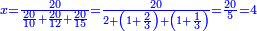
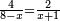
![\scriptstyle{\color{blue}{x=\left[\left[\left(8-x\right)+\left(x+1\right)\right]\sdot\frac{2}{2+4}\right]-1=\left(9\sdot\frac{2}{6}\right)-1=\left(9\sdot\frac{1}{3}\right)-1=2}}](/mediawiki/images/math/d/4/2/d42b90936bb2eba7513ff2d1773924bb.png)

![{\color{blue}{\begin{align}\scriptstyle x&\scriptstyle=\left[\left[\left(x-1\right)+\left(10-x\right)\right]\sdot\frac{3}{3+5}\right]+1=\left(9\sdot\frac{3}{8}\right)+1=\left[9\sdot\left[\frac{1}{4}+\left(\frac{1}{2}\sdot\frac{1}{4}\right)\right]\right]+1=\left(3+\frac{3}{8}\right)+1\\&\scriptstyle=4+\frac{3}{8}\\\end{align}}}](/mediawiki/images/math/d/e/a/dea0e61a0b9149869024b1b1ae347ab5.png)
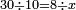
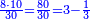
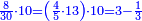
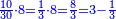
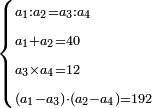

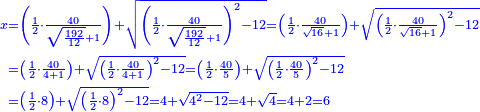

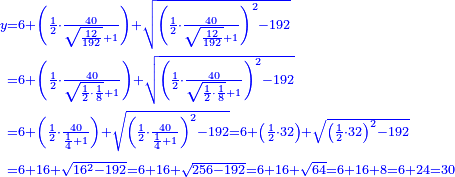


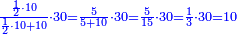
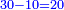
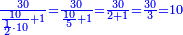
![\scriptstyle\left(x\sdot\frac{10}{30}\right)-\left[\left(30-x\right)\sdot\frac{5}{30}\right]=1](/mediawiki/images/math/3/4/6/346922a7313f2cbdbdec4b3250f5981e.png)
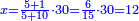
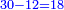

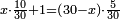


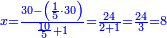
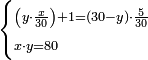
![\scriptstyle{\color{blue}{y=\frac{\left[\left(5-1\right)\sdot30\right]-80}{5}=\frac{\left(4\sdot30\right)-80}{5}=\frac{40}{5}=8}}](/mediawiki/images/math/0/4/e/04ef367b12d05fc6cb75960e54fe97bd.png)
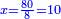

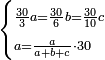
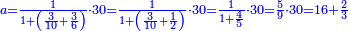
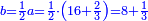

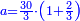
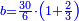
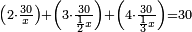
![\scriptstyle{\color{blue}{x=\frac{\left(2\sdot30\right)+\left[\left(3\sdot30\right)\sdot2\right]+\left[\left(4\sdot30\right)\sdot3\right]}{30}=\frac{60+180+360}{30}=\frac{600}{30}=20}}](/mediawiki/images/math/5/6/2/562fda47469492be1aa79eec119908b6.png)
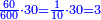
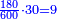
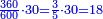
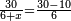

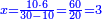
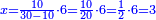
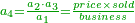


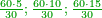

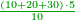



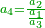

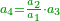



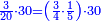
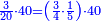



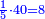


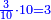
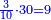

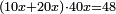
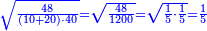
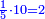
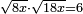
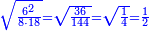

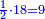
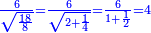
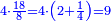


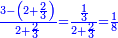
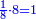
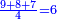




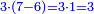
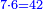
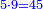
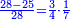
![\scriptstyle{\color{blue}{a=42-\left[\left(\frac{3}{4}\sdot\frac{1}{7}\right)\sdot42\right]=37+\frac{1}{2}}}](/mediawiki/images/math/8/a/4/8a4cc703188e80aec577434c60184cdb.png)
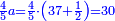


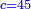

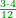
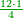

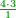
![\scriptstyle\left(2x\sdot6\right)-\left[x\sdot\left(1+2\right)\right]=6-4](/mediawiki/images/math/a/1/a/a1a3080a03d2b52fc41377b632dd56a1.png)
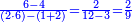
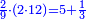
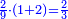
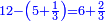
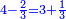
![\scriptstyle\left[x\sdot\left(5+7\right)\right]-\left(2x\sdot1\right)=4-1](/mediawiki/images/math/a/3/b/a3b4865ffc423e382f8577a723762bdc.png)
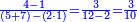
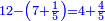
![\scriptstyle{\color{blue}{4-\left[3+\frac{1}{2}+\left(\frac{1}{2}\sdot\frac{1}{5}\right)\right]=\frac{2}{5}}}](/mediawiki/images/math/8/a/a/8aa11d0bf6e1875a1242afc9dac56e76.png)
![\scriptstyle\left(2x\sdot6\right)-\left[x\sdot\left[\frac{3}{4}+\left(1+\frac{1}{4}\right)\right]\right]=6-4](/mediawiki/images/math/c/8/0/c8067ad9007f3a7cda8edbd70f832bbe.png)
![\scriptstyle{\color{blue}{\frac{6-4}{\left(2\sdot6\right)-\left[\frac{3}{4}+\left(1+\frac{1}{4}\right)\right]}=\frac{2}{12-2}=\frac{2}{10}=\frac{1}{5}}}](/mediawiki/images/math/f/7/1/f7120260ad540faf61afc12a17ca71a6.png)
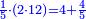
![\scriptstyle{\color{blue}{\frac{1}{5}\sdot\left[\frac{3}{4}+\left(1+\frac{1}{4}\right)\right]=\frac{2}{5}}}](/mediawiki/images/math/2/b/3/2b3267957a45ab5a0e4f837c40f745c6.png)
![\scriptstyle\left[x\sdot\left[\left(5+\frac{1}{4}\right)+\left(6+\frac{3}{4}\right)\right]\right]-\left(2x\sdot2\right)=4-2](/mediawiki/images/math/3/4/5/345afae745fd9cc75c453b9aabbf4fe1.png)
![\scriptstyle{\color{blue}{\frac{4-2}{\left[\left(5+\frac{1}{4}\right)+\left(6+\frac{3}{4}\right)\right]-\left(2\sdot2\right)}=\frac{2}{12-4}=\frac{2}{8}=\frac{1}{4}}}](/mediawiki/images/math/e/9/7/e97cddba5666298a7ab4407e7bfb239f.png)
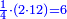
![\scriptstyle{\color{blue}{\frac{1}{4}\sdot\left[\left(5+\frac{1}{4}\right)+\left(6+\frac{3}{4}\right)\right]=3}}](/mediawiki/images/math/8/2/6/826bece0bd15376a8f55506f242f8dc6.png)

![\scriptstyle\left(3x\sdot5\right)-\left[x\sdot\left[\frac{1}{4}+\frac{1}{2}+\left(1+\frac{1}{4}\right)\right]\right]=5-4](/mediawiki/images/math/4/e/b/4eb0927ce361b75f8b3baf535c21318f.png)
![\scriptstyle{\color{blue}{\frac{5-4}{\left(3\sdot5\right)-\left[\frac{1}{4}+\frac{1}{2}+\left(1+\frac{1}{4}\right)\right]}=\frac{1}{15-2}=\frac{1}{13}}}](/mediawiki/images/math/9/c/5/9c5c95b523f39c1b26e6b5856cb86e3b.png)
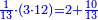
![\scriptstyle{\color{blue}{\frac{1}{13}\sdot\left[\frac{1}{4}+\frac{1}{2}+\left(1+\frac{1}{4}\right)\right]=\frac{2}{13}}}](/mediawiki/images/math/4/2/3/423de9f88d80f84b155e54b8772e55f8.png)
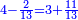
![\scriptstyle\left[x\sdot\left[\left(5+\frac{1}{4}\right)+\left(6+\frac{1}{2}\right)+\left(7+\frac{1}{4}\right)\right]\right]-\left(3x\sdot3\right)=4-3](/mediawiki/images/math/f/5/d/f5d280babd1c03df9b2ee340c2f82645.png)
![\scriptstyle{\color{blue}{\frac{4-3}{\left[\left(5+\frac{1}{4}\right)+\left(6+\frac{1}{2}\right)+\left(7+\frac{1}{4}\right)\right]-\left(3\sdot3\right)}=\frac{1}{19-9}=\frac{1}{10}}}](/mediawiki/images/math/d/b/9/db95614cdb6ceb7cc5861b1397207e39.png)

![\scriptstyle{\color{blue}{4-\left[\frac{1}{10}\sdot\left[\left(5+\frac{1}{4}\right)+\left(6+\frac{1}{2}\right)+\left(7+\frac{1}{4}\right)\right]\right]=2+\left(\frac{1}{2}\sdot\frac{1}{5}\right)}}](/mediawiki/images/math/4/b/d/4bd6a1017a84c6d09562d1901ef0ae2c.png)
![\scriptstyle\left[4x\sdot\left(5+\frac{3}{4}\right)\right]-\left[x\sdot\left[\frac{1}{2}+\frac{3}{4}+\left(2+\frac{1}{2}\right)+\left(3+\frac{1}{4}\right)\right]\right]=\left(5+\frac{3}{4}\right)-\left(3+\frac{3}{4}\right)](/mediawiki/images/math/6/2/8/6281af632a6c43529673337ff9e95a22.png)
![\scriptstyle{\color{blue}{x=\frac{\left(5+\frac{3}{4}\right)-\left(3+\frac{3}{4}\right)}{\left[4\sdot\left(5+\frac{3}{4}\right)\right]-\left[\frac{1}{2}+\frac{3}{4}+\left(2+\frac{1}{2}\right)+\left(3+\frac{1}{4}\right)\right]}=\frac{2}{23-7}=\frac{2}{16}=\frac{1}{8}}}](/mediawiki/images/math/d/e/d/ded43f5fb915555a080b1de4f9c1ef4e.png)

![\scriptstyle{\color{blue}{\frac{1}{8}\sdot\left[\frac{1}{2}+\frac{3}{4}+\left(2+\frac{1}{2}\right)+\left(3+\frac{1}{4}\right)\right]=\frac{7}{8}}}](/mediawiki/images/math/1/2/c/12cc4230f3ecd1287b595695b3141c40.png)
![\scriptstyle{\color{blue}{\frac{1}{8}\sdot\left[4\sdot\left(5+\frac{3}{4}\right)\right]=2+\frac{7}{8}}}](/mediawiki/images/math/1/9/3/193a763fd916da00b03ce872ab8db939.png)
![\scriptstyle4x\sdot12-\left[x\sdot\left[\frac{3}{4}+\left(1+\frac{1}{4}\right)+\left(1+\frac{1}{2}\right)+\left(2+\frac{1}{2}\right)\right]\right]=12-4](/mediawiki/images/math/1/0/b/10b5b7964f1a5a51a2d28692a15d7481.png)
![\scriptstyle{\color{blue}{x=\frac{12-4}{\left(4\sdot12\right)-\left[\frac{3}{4}+\left(1+\frac{1}{4}\right)+\left(1+\frac{1}{2}\right)+\left(2+\frac{1}{2}\right)\right]}=\frac{8}{48-6}=\frac{8}{42}=\frac{1}{7}+\left(\frac{1}{3}\sdot\frac{1}{7}\right)}}](/mediawiki/images/math/b/8/2/b82678cfe92167e0c8be52f20bc23f81.png)
![\scriptstyle{\color{blue}{\left[\frac{1}{7}+\left(\frac{1}{3}\sdot\frac{1}{7}\right)\right]\sdot\left(4\sdot12\right)=9+\frac{1}{7}}}](/mediawiki/images/math/c/8/7/c87d62c86ac0cbd46985e02ee0f8b6fd.png)
![\scriptstyle{\color{blue}{\left[\frac{1}{7}+\left(\frac{1}{3}\sdot\frac{1}{7}\right)\right]\sdot\left[\frac{3}{4}+\left(1+\frac{1}{4}\right)+\left(1+\frac{1}{2}\right)+\left(2+\frac{1}{2}\right)\right]=1+\frac{1}{7}}}](/mediawiki/images/math/f/8/9/f89fe031ec446389c11a11681d8da0c7.png)
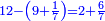
![\scriptstyle4x\sdot12-\left[x\sdot\left[\left[12-\left(8+\frac{1}{2}\right)\right]+\left[12-\left(7+\frac{3}{4}\right)\right]+\left[12-\left(6+\frac{1}{2}\right)\right]+\left[12-\left(5+\frac{1}{4}\right)\right]\right]\right]=12-\left(12-4\right)](/mediawiki/images/math/1/7/6/176fc78a09bb823d327cff56957f2455.png)
![\scriptstyle4x\sdot12-\left[x\sdot\left[\left(3+\frac{1}{2}\right)+\left(4+\frac{1}{4}\right)+\left(5+\frac{1}{2}\right)+\left(6+\frac{3}{4}\right)\right]\right]=12-8](/mediawiki/images/math/f/f/b/ffb05c32fd2cc4ec70ac2fac0ff9c0e8.png)
![\scriptstyle{\color{blue}{x=\frac{12-8}{\left(4\sdot12\right)-\left[\left(3+\frac{1}{2}\right)+\left(4+\frac{1}{4}\right)+\left(5+\frac{1}{2}\right)+\left(6+\frac{3}{4}\right)\right]}=\frac{4}{48-20}=\frac{4}{28}=\frac{1}{7}}}](/mediawiki/images/math/f/2/b/f2b727fa41789725a2d1854914de2dd6.png)
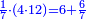
![\scriptstyle{\color{blue}{\frac{1}{7}\sdot\left[\left(3+\frac{1}{2}\right)+\left(4+\frac{1}{4}\right)+\left(5+\frac{1}{2}\right)+\left(6+\frac{3}{4}\right)\right]=2+\frac{6}{7}}}](/mediawiki/images/math/b/e/6/be6152bd21ff14f9ac2f4d5a12f57463.png)
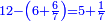
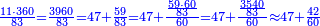
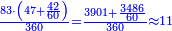
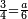
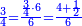
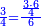
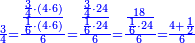
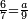
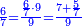
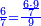
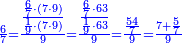

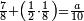
![\scriptstyle{\color{blue}{\frac{7}{8}+\left(\frac{1}{2}\sdot\frac{1}{8}\right)=\frac{\left[\frac{7}{8}+\left(\frac{1}{2}\sdot\frac{1}{8}\right)\right]\sdot10}{10}=\frac{9}{10}+\left(\frac{3}{8}\sdot\frac{1}{10}\right)}}](/mediawiki/images/math/6/2/0/620d0016d0da06ef18a40d3d9a967edd.png)
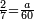

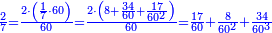


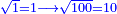
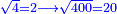
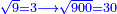



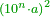
![\scriptstyle x^2=\left(10a\right)^2+\left[2\sdot\left(10a\right)\right]+1\longrightarrow x=10a+1](/mediawiki/images/math/5/b/0/5b0eda3626f16173640aa6488bdcfe12.png)
![\scriptstyle x^2=\left(10a\right)^2-\left[2\sdot\left(10a\right)\right]+1\longrightarrow x=10a-1](/mediawiki/images/math/6/9/1/6914ed525410bbc811bfc1b814c7c88f.png)
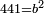

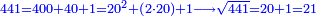
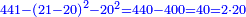

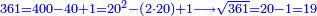
![\scriptstyle{\color{blue}{20^2-\left[361-\left(20-19\right)^2\right]=400-360=40=2\sdot20}}](/mediawiki/images/math/c/2/c/c2c27f2d1246b8105b3326c2e2bb0d87.png)
![\scriptstyle x^2=\left(10a\right)^2+\left[2\sdot2\left(10a\right)\right]+4\longrightarrow x=10a+2](/mediawiki/images/math/1/0/2/102706261cd117d159b785e25fe58d62.png)
![\scriptstyle x^2=\left(10a\right)^2-\left[2\sdot2\left(10a\right)\right]+4\longrightarrow x=10a-2](/mediawiki/images/math/d/f/b/dfba453db89d6e06469b7751ec78ae03.png)
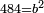

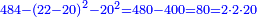

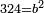

![\scriptstyle{\color{blue}{20^2-\left[324-\left(20-18\right)^2\right]=400-320=80=2\sdot2\sdot20}}](/mediawiki/images/math/e/9/5/e95964a1fed2285c919ecc9238d3ab87.png)

![\scriptstyle x^2=\left(10a\right)^2+\left[2\sdot3\left(10a\right)\right]+9\longrightarrow x=10a+3](/mediawiki/images/math/1/0/4/10433c10e3e288329f1a6c2244b1f53d.png)
![\scriptstyle x^2=\left(10a\right)^2-\left[2\sdot3\left(10a\right)\right]+9\longrightarrow x=10a-3](/mediawiki/images/math/f/f/6/ff6f80a51eba80c1c4ffcbceaa663ece.png)
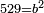
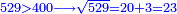
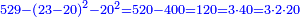
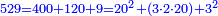
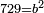
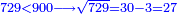
![\scriptstyle{\color{blue}{30^2-\left[729-\left(30-27\right)^2\right]=900-720=180=3\sdot2\sdot30}}](/mediawiki/images/math/c/c/1/cc17220e49784d7225993f7962ed1324.png)
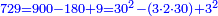
![\scriptstyle\left(10a+5\right)^2=\left[\left[10\sdot\left(a+1\right)\right]-5\right]^2](/mediawiki/images/math/5/7/9/579b8a5f72ed4b0997c92a0b11930f24.png)
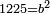
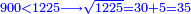

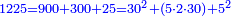

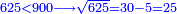
![\scriptstyle{\color{blue}{30^2-\left[625-\left(30-25\right)^2\right]=900-600=300=5\sdot2\sdot30}}](/mediawiki/images/math/3/7/3/373b859db08c1448562e0332e897e6e9.png)
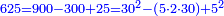
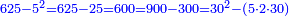
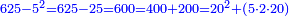
![\scriptstyle{\color{blue}{\left[\left(2\sdot10\right)+5\right]^2=\left(20+5\right)^2=625=\left(30-5\right)^2=\left[\left(3\sdot10\right)-5\right]^2}}](/mediawiki/images/math/9/1/2/9129b10118885049df632bd2d40d63ae.png)
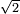
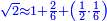
![\scriptstyle{\color{blue}{\sqrt{2}\approx1+\frac{2}{6}+\left(\frac{1}{2}\sdot\frac{1}{6}\right)-\frac{\left(\frac{1}{2}\sdot\frac{1}{6}\right)^2}{2\sdot\left[1+\frac{2}{6}+\left(\frac{1}{2}\sdot\frac{1}{6}\right)\right]}}}](/mediawiki/images/math/0/2/a/02a5749a5be75d8750bee2f6ca4401f2.png)
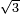


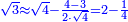
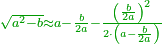

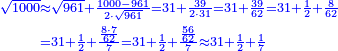
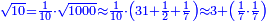
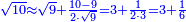
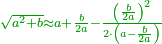


![\scriptstyle{\color{blue}{\sqrt{\frac{6}{8}+\left(\frac{1}{8}\sdot\frac{1}{8}\right)}=\frac{1}{8}\sdot\sqrt{8^2\sdot\left[\frac{6}{8}+\left(\frac{1}{8}\sdot\frac{1}{8}\right)\right]}=\frac{1}{8}\sdot\sqrt{64\sdot\left[\frac{6}{8}+\left(\frac{1}{8}\sdot\frac{1}{8}\right)\right]}=\frac{1}{8}\sdot\sqrt{49}=\frac{7}{8}}}](/mediawiki/images/math/a/d/1/ad1a1b4f26ded493b5b94fda2c99b55c.png)
![\scriptstyle{\color{blue}{\sqrt{\frac{6}{8}+\left(\frac{1}{8}\sdot\frac{1}{8}\right)}=\frac{1}{2}\sdot\sqrt{2^2\sdot\left[\frac{6}{8}+\left(\frac{1}{8}\sdot\frac{1}{8}\right)\right]}=\frac{1}{2}\sdot\sqrt{3+\left(\frac{1}{2}\sdot\frac{1}{8}\right)}=\frac{1}{2}\sdot\left(1+\frac{3}{4}\right)=\frac{7}{8}}}](/mediawiki/images/math/1/d/f/1df6109380b8df4b9dc4c8c6154e409b.png)
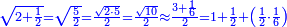

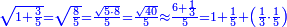
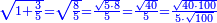
 and the root of fourths are seconds
and the root of fourths are seconds 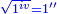 .
.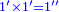
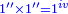
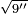
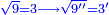
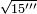
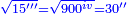
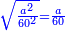

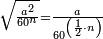
![\scriptstyle{\color{OliveGreen}{\sqrt{a^2+\frac{\left(2\sdot a\sdot n\right)+m}{60}}=\sqrt{\left(a+\frac{n}{60}\right)^2+\left[\frac{m}{60}-\left(\frac{n}{60}\right)^2\right]}\approx a+\frac{n}{60}}}](/mediawiki/images/math/b/9/8/b985ba34fc5df8c0513442bca361c372.png)
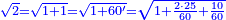
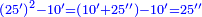
![{\color{blue}{\begin{align}\scriptstyle\sqrt{2}&\scriptstyle=\sqrt{1+1}=\sqrt{1+60^\prime}=\sqrt{1+\frac{2\sdot24}{60}+12^\prime}=\sqrt{\left(1+24^\prime\right)^2+\left[12^\prime-\left(24^\prime\right)^2\right]}\\&\scriptstyle=\sqrt{\left(1+24^\prime\right)^2+\left[12^\prime-\left(9^\prime+36^{\prime\prime}\right)\right]}=\sqrt{\left(1+24^\prime\right)^2+\left(2^\prime+24^{\prime\prime}\right)}\approx1+24^\prime\\\end{align}}}](/mediawiki/images/math/1/9/a/19a5e62bfc43b5d1333713069849f88d.png)
![{\color{blue}{\begin{align}\scriptstyle\sqrt{2}&\scriptstyle=\sqrt{\left(1+24^\prime\right)^2+\left(2^\prime+24^{\prime\prime}\right)}=\sqrt{\left(1+24^\prime\right)^2+\left(\frac{168\sdot51}{60^3}+72^{\prime\prime\prime}\right)}\\&\scriptstyle=\sqrt{\left(1+24^\prime\right)^2+\left[\left[2\sdot\left(1+24^\prime\right)\sdot51^{\prime\prime}\right]+72^{\prime\prime\prime}\right]}=\sqrt{\left(1+24^\prime+51^{\prime\prime}\right)^2+\left[72^{\prime\prime\prime}-\left(51^{\prime\prime}\right)^2\right]}\\&\scriptstyle=\sqrt{\left(1+24^\prime+51^{\prime\prime}\right)^2+\left[72^{\prime\prime\prime}-\left(43^{\prime\prime}+21^{\prime\prime\prime\prime}\right)\right]}\\&\scriptstyle=\sqrt{\left(1+24^\prime+51^{\prime\prime}\right)^2+\left(28^{\prime\prime\prime}+39^{\prime\prime\prime\prime}\right)}\approx1+24^\prime+51^{\prime\prime}\\\end{align}}}](/mediawiki/images/math/6/1/e/61e5dc28b8cf5063a933a4f80db978b3.png)
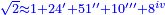
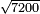
![{\color{blue}{\begin{align}\scriptstyle\sqrt{7200}&\scriptstyle=\sqrt{2\sdot3600}=\sqrt{2\sdot60^2}=60\sdot\sqrt{2}\approx60\sdot\left(1+24^\prime+51^{\prime\prime}+\ldots\right)\\&\scriptstyle=60\sdot\left[\left(60+24\right)^\prime+51^{\prime\prime}+\ldots\right]=60\sdot\left(84^\prime+51^{\prime\prime}+\ldots\right)=84+51^\prime+\ldots\\\end{align}}}](/mediawiki/images/math/d/d/f/ddf4daa2baeb054cf4ce7b82841458e0.png)
![\scriptstyle{\color{OliveGreen}{\frac{b}{2a}>30^\prime\longrightarrow\left[\left(a+1\right)^2-\left(a^2+b\right)\right]<\left(a^2+b\right)-a^2}}](/mediawiki/images/math/2/0/0/200d3db83eaf9d79f5857f2b89703dff.png)
![\scriptstyle{\color{OliveGreen}{\frac{b}{2a}<30^\prime\longrightarrow\left[\left(a+1\right)^2-\left(a^2+b\right)\right]>\left(a^2+b\right)-a^2}}](/mediawiki/images/math/0/e/8/0e8535ccaa17614915593b7f3491cc60.png)



![\scriptstyle{\color{OliveGreen}{\sqrt{a^2+b}\approx a+\frac{b}{2a}-\frac{\left(\frac{b}{2a}\right)^2}{2\sdot\left(a+\frac{b}{2a}\right)}-\frac{\left[\frac{\left(\frac{b}{2a}\right)^2}{2\sdot\left(a+\frac{b}{2a}\right)}\right]^2}{2\sdot\left[a+\frac{b}{2a}-\frac{\left(\frac{b}{2a}\right)^2}{2\sdot\left(a+\frac{b}{2a}\right)}\right]}}}](/mediawiki/images/math/8/9/6/8966db37705ce5b03d2f73262fe412d1.png)