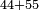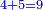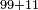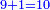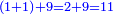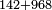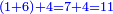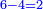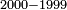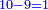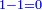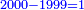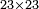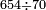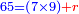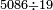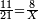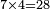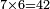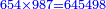Difference between revisions of "ספר אגריס"
From mispar
(→Multiplication) |
(→Appendix: Bibliography) |
||
| (71 intermediate revisions by the same user not shown) | |||
| Line 1: | Line 1: | ||
| + | {{#annotpage: time="1300-1400", peshat_title="00002045"}} | ||
{| | {| | ||
|- | |- | ||
| Line 29: | Line 30: | ||
| | | | ||
|- | |- | ||
| + | !The numerals | ||
| | | | ||
| − | + | |- | |
| − | + | |The nine numerals - the nine first Hebrew letters | |
| + | |style="text-align:right;"|ומהנה אתחיל ואומר ה{{#annot:term|204,1332|CMl9}}אותיות{{#annotend:CMl9}} היוצאות במילת אגריס לפועל הם ט' כמספרם ואילו הם | ||
| + | |- | ||
| + | | 1 2 3 4 5 6 7 8 9 | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
|- | |- | ||
| − | | | + | |ט||ח||ז||ו||ה||ד||ג||ב||א |
| − | | | + | |} |
| + | |- | ||
| + | |} | ||
|- | |- | ||
|Every number is written with these nine numerals | |Every number is written with these nine numerals | ||
|style="text-align:right;"|ובהם כתוב כל מספר | |style="text-align:right;"|ובהם כתוב כל מספר | ||
|- | |- | ||
| + | !The written ranks [= decimal places] | ||
| | | | ||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| − | + | !Units | |
| − | + | |style="text-align:right;"|ודע כל מניין שתחפוץ לכתוב מא' עד ט' | |
| − | |style="text-align:right;"|ודע כל מניין שתחפוץ לכתוב מא' עד ט' תוכל לכתוב באות אחת כגון אחת | + | |- |
| + | |written with one letter only | ||
| + | |style="text-align:right;"|תוכל לכתוב באות אחת כגון אחת א' או שתים ב' כו' עד ט‫' | ||
|- | |- | ||
|Ten cannot be indicated by the nine numerals | |Ten cannot be indicated by the nine numerals | ||
| Line 53: | Line 64: | ||
|- | |- | ||
|Zero: 10 - the zero indicates that 1 is in the second rank; 1 without a zero is one | |Zero: 10 - the zero indicates that 1 is in the second rank; 1 without a zero is one | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וה{{#annot:term|205,1472|8fnF}}גלגל{{#annotend:8fnF}} יורה שהא' במעלה שנייה והיא עשרה והא' בלא גלגל אחד |
|- | |- | ||
| − | + | !Tens | |
| − | |||
|style="text-align:right;"|וכן כל האותיות עד ט‫' | |style="text-align:right;"|וכן כל האותיות עד ט‫' | ||
|- | |- | ||
| Line 64: | Line 74: | ||
|- | |- | ||
| | | | ||
| − | + | *20 | |
|style="text-align:right;"|וכדרך זה תכתוב אם דעתך לכתוב עשרים תכתוב 0"ב | |style="text-align:right;"|וכדרך זה תכתוב אם דעתך לכתוב עשרים תכתוב 0"ב | ||
|- | |- | ||
| | | | ||
| − | + | *30 | |
|style="text-align:right;"|שלשים 0"ג | |style="text-align:right;"|שלשים 0"ג | ||
|- | |- | ||
| | | | ||
| − | + | *40 | |
|style="text-align:right;"|ארבעים 0"ד | |style="text-align:right;"|ארבעים 0"ד | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|ועל דרך זה תוכל להבין כל אות שהיא | + | |style="text-align:right;"|ועל דרך זה תוכל להבין כל אות שהיא ב{{#annot:term|203,1316|38lN}}מעלה {{#annotend:38lN}}שנייה שהיא עשירית |
|- | |- | ||
| | | | ||
| − | + | *90 | |
|style="text-align:right;"|וכדרך זה תכתוב עד תשעים והוא 0"ט | |style="text-align:right;"|וכדרך זה תכתוב עד תשעים והוא 0"ט | ||
| + | |- | ||
| + | !Hundreds | ||
| + | | | ||
|- | |- | ||
| | | | ||
| − | + | *100 | |
| − | |||
|style="text-align:right;"|אך מאה היאך תרצה לכתוב תכתוב א' במעלה השלישית כזה 00"א | |style="text-align:right;"|אך מאה היאך תרצה לכתוב תכתוב א' במעלה השלישית כזה 00"א | ||
|- | |- | ||
| | | | ||
| − | + | *200 | |
|style="text-align:right;"|ב' מאות כזה 00"ב | |style="text-align:right;"|ב' מאות כזה 00"ב | ||
|- | |- | ||
| | | | ||
| − | + | *300 | |
|style="text-align:right;"|ג' מאות 00"ג | |style="text-align:right;"|ג' מאות 00"ג | ||
|- | |- | ||
| | | | ||
| − | + | *400 | |
|style="text-align:right;"|ד' מאות 00"ד | |style="text-align:right;"|ד' מאות 00"ד | ||
|- | |- | ||
| | | | ||
| − | + | *900 | |
|style="text-align:right;"|וכן תעשה עד ט' מאות כזה 00"ט | |style="text-align:right;"|וכן תעשה עד ט' מאות כזה 00"ט | ||
|- | |- | ||
| + | !Thousands | ||
| | | | ||
| − | + | |- | |
| − | + | | | |
| + | *1000 | ||
|style="text-align:right;"|רק אלף היאך תרצה לכתוב תכתוב במעלה רביעית כזה 000"א | |style="text-align:right;"|רק אלף היאך תרצה לכתוב תכתוב במעלה רביעית כזה 000"א | ||
|- | |- | ||
| | | | ||
| − | + | *2000 | |
|style="text-align:right;"|אלפים כזה 000"ב | |style="text-align:right;"|אלפים כזה 000"ב | ||
|- | |- | ||
| | | | ||
| − | + | *3000 | |
|style="text-align:right;"|ג' אלפים כזה 000"ג | |style="text-align:right;"|ג' אלפים כזה 000"ג | ||
|- | |- | ||
| | | | ||
| − | + | *4000 | |
|style="text-align:right;"|ד' אלפים 000"ד | |style="text-align:right;"|ד' אלפים 000"ד | ||
|- | |- | ||
| | | | ||
| − | + | *9000 | |
|style="text-align:right;"|וכו' עד ט' אלפים כזה 000"ט | |style="text-align:right;"|וכו' עד ט' אלפים כזה 000"ט | ||
|- | |- | ||
| + | !Tens of thousands | ||
| | | | ||
| − | + | |- | |
| − | + | | | |
| + | *10000 | ||
|style="text-align:right;"|ועשרת אלפים תכתוב כזה 0000"א והא' עומד במעלה החמישית | |style="text-align:right;"|ועשרת אלפים תכתוב כזה 0000"א והא' עומד במעלה החמישית | ||
|- | |- | ||
| | | | ||
| − | + | *20000 | |
|style="text-align:right;"|עשרים אלפים 0000"ב | |style="text-align:right;"|עשרים אלפים 0000"ב | ||
|- | |- | ||
| | | | ||
| − | + | *30000 | |
|style="text-align:right;"|שלשים אלפים 0000"ג | |style="text-align:right;"|שלשים אלפים 0000"ג | ||
|- | |- | ||
| | | | ||
| − | + | *40000 | |
|style="text-align:right;"|ארבעים אלפים כזה 0000"ד | |style="text-align:right;"|ארבעים אלפים כזה 0000"ד | ||
|- | |- | ||
| | | | ||
| − | + | *90000 | |
|style="text-align:right;"|וכו' עד תשעים אלפים כזה 0000"ט | |style="text-align:right;"|וכו' עד תשעים אלפים כזה 0000"ט | ||
|- | |- | ||
| + | !Hundreds of thousands | ||
| | | | ||
| − | * | + | |- |
| + | | | ||
| + | *100000, etc. | ||
|style="text-align:right;"|מאה אלפי' תכתוב הא' במעלה שישית כזה 00000"א וכו‫' | |style="text-align:right;"|מאה אלפי' תכתוב הא' במעלה שישית כזה 00000"א וכו‫' | ||
|- | |- | ||
| Line 163: | Line 182: | ||
== Addition == | == Addition == | ||
| − | + | |style="text-align:right;"|<big>שער ה{{#annot:term|154,1208|JhNc}}חיבור{{#annotend:JhNc}}</big> | |
| + | |- | ||
| + | |To let you know how to sum two numbers together. | ||
| + | |style="text-align:right;"|להודיעך איך תתחבר שני חשבונות יחד | ||
| + | |- | ||
| + | |Write one number in a line and beneath is the second number: units corresponding to units and tens corresponding to tens, meaning write the digit [lit. letter] that is in the first rank beneath the digit that is also in the first rank, then the second beneath the second in rank and so on. | ||
| + | |style="text-align:right;"|תכתוב חשבון אחד בשורה ותחתיו חשבון השני פרט נגד פרט וכלל נגד כלל פי' ה{{#annot:term|204,1332|vv7v}}אות{{#annotend:vv7v}} שהיא במעלה הראשונה כתוב האות תחת אות שהיא ג"כ למעלה ראשונה והשנייה תחת השנייה במעלה וכן כולם | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:44+55|154|HyDz}}Example: you wish to add 44 to 55. | ||
| + | :<math>\scriptstyle44+55</math> | ||
| + | |style="text-align:right;"|דומיון רצונך לחבר ד' וארבעים לה' וחמשים{{#annotend:HyDz}} | ||
| + | |- | ||
| + | | | ||
| + | :Write as follows: | ||
| + | |style="text-align:right;"|תכתוב כך | ||
| + | |- | ||
| + | | | ||
| + | :{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |4||4 | ||
| + | |- | ||
| + | |5||5 | ||
| + | |} | ||
|- | |- | ||
| + | |} | ||
| | | | ||
| − | |style="text-align: | + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" |
|- | |- | ||
| | | | ||
| − | |||
| − | |||
{|style="margin-left: auto; margin-right: 0px;" | {|style="margin-left: auto; margin-right: 0px;" | ||
|- | |- | ||
| − | + | |ד||ד | |
|- | |- | ||
| − | + | |ה||ה | |
|} | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | :*Sum 4 with 5 in the bottom [row], it is 9. <math>\scriptstyle{\color{blue}{4+5=9}}</math> | ||
| + | |style="text-align:right;"|חבר ד' עם ה' שבתחתון והיא ט‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*Sum the second 4 with the second 5, it is 9. <math>\scriptstyle{\color{blue}{4+5=9}}</math> |
| + | |style="text-align:right;"|חביר ד' שנייה ה' שנייה והוא ט‫' | ||
| + | |- | ||
| + | | | ||
| + | :Thus, we found that their sum is 99, which is ninty-nine. | ||
| + | :<math>\scriptstyle{\color{blue}{44+55=99}}</math> | ||
| + | |style="text-align:right;"|א"כ מצאנו {{#annot:term|388,1678|oJpi}}צירופם{{#annotend:oJpi}} ט"ט והם תשעה ותשעים | ||
| + | |- | ||
| + | |Sum all the digits according to this way. | ||
| + | |style="text-align:right;"|ובזה הדרך חבר כל האותיות | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:99+11|154|my7M}}Another example: | ||
| + | :<math>\scriptstyle99+11</math> | ||
| + | |style="text-align:right;"|דומיון אחר{{#annotend:my7M}} | ||
| + | |- | ||
| + | | | ||
| + | :{|class="wikitable" style="color:blue; text-align:center;" | ||
|- | |- | ||
| | | | ||
| − | |||
| − | |||
{|style="margin-left: auto; margin-right: 0px;" | {|style="margin-left: auto; margin-right: 0px;" | ||
|- | |- | ||
| − | | | + | |9||9 |
|- | |- | ||
| − | | | + | |1||1 |
|} | |} | ||
|- | |- | ||
| + | |} | ||
| | | | ||
| − | |style="text-align: | + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" |
|- | |- | ||
| | | | ||
| − | |||
{|style="margin-left: auto; margin-right: 0px;" | {|style="margin-left: auto; margin-right: 0px;" | ||
|- | |- | ||
| − | | | + | |ט||ט |
|- | |- | ||
| − | | | + | |א||א |
|} | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | :*Add 9 to 1 that is on the bottom [row], which is 11, [it is] ten. <math>\scriptstyle{\color{blue}{9+1=10}}</math> | ||
| + | |style="text-align:right;"|{{#annot:term|178,1165|4Gks}}חבר{{#annotend:4Gks}} ט' על א' שבתחתה והיא א"א עשרה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|חבר ב' על ח' זה עשרה כתוב 0' והא' הנותרת חבר עם הו' ויהיה ז | + | ::Write 0 and you are left with 1, which is in the second rank. |
| + | |style="text-align:right;"|כתוב 0' ישאר לך א' והיא א' שנייה במעלה | ||
| + | |- | ||
| + | | | ||
| + | :*Add it to the second 1 that is also in the [second] rank, it is 2. Add the 2 to the 9 in the second rank, it is 11. <math>\scriptstyle{\color{blue}{\left(1+1\right)+9=2+9=11}}</math> | ||
| + | |style="text-align:right;"|{{#annot:term|178,1810|UT9N}}צרוף{{#annotend:UT9N}} אותה במעלה לא' השנייה גם כן במעלה ויהיה ב' צרוף הב' על הט' במעלה שנייה ויהיה א"א | ||
| + | |- | ||
| + | | | ||
| + | :And 0 first, it is 110. | ||
| + | :<math>\scriptstyle{\color{blue}{99+11=110}}</math> | ||
| + | |style="text-align:right;"|ובתחילה 0' ויהיה 0'א'א‫' | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:142+968|154|EWxV}}Another example: | ||
| + | :<math>\scriptstyle142+968</math> | ||
| + | |style="text-align:right;"|דומיון אחר{{#annotend:EWxV}} | ||
| + | |- | ||
| + | | | ||
| + | :{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |1||4||2 | ||
| + | |- | ||
| + | |9||6||8 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |א||ד||ב | ||
| + | |- | ||
| + | |ט||ו||ח | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | :*Add 2 to 8, it is ten. <math>\scriptstyle{\color{blue}{2+8=10}}</math> | ||
| + | |style="text-align:right;"|חבר ב' על ח' זה עשרה | ||
| + | |- | ||
| + | | | ||
| + | ::Write 0. | ||
| + | |style="text-align:right;"|כתוב 0‫' | ||
| + | |- | ||
| + | | | ||
| + | :*Sum the remaining 1 with 6, it is 7. Add it to 4, it is 11. <math>\scriptstyle{\color{blue}{\left(1+6\right)+4=7+4=11}}</math> | ||
| + | |style="text-align:right;"|והא' הנותרת חבר עם הו' ויהיה ז' חברה על הד' ויהיה א"א | ||
| + | |- | ||
| + | | | ||
| + | ::Write 1 in the second rank. | ||
| + | |style="text-align:right;"|כתוב א' במעלה שנייה | ||
| + | |- | ||
| + | | | ||
| + | :*Add 1 in the third rank to 9 and 1, it is 11. <math>\scriptstyle{\color{blue}{1+9+1=11}}</math> | ||
| + | |style="text-align:right;"|והא' השלישי' במעלה חברה על הט' ועל הא' זהו א"א | ||
| + | |- | ||
| + | | | ||
| + | :So it is 1110. | ||
| + | :<math>\scriptstyle{\color{blue}{142+968=1110}}</math> | ||
| + | |style="text-align:right;"|אז הוא 0אא"א | ||
|- | |- | ||
|} | |} | ||
| Line 213: | Line 349: | ||
== Subtraction == | == Subtraction == | ||
| − | + | |style="text-align:right;"|<big>שער ה{{#annot:term|155,1657|qLXy}}חסור{{#annotend:qLXy}}</big> | |
|- | |- | ||
| + | |If you know a number and you wish to subtract [from it] a certain amount and know what remains from the first number after subtracting the amount from it, I will teach you this: | ||
| + | |style="text-align:right;"|אם ידעת חשבון ורצונך {{#annot:term|181,1362|Veye}}לחסר{{#annotend:Veye}} מי"ט סך אחד וחפצתה לידע מה יסיר מן החשבון הראשון אחר {{#annot:term|155,2268|VrLL}}סלוק{{#annotend:VrLL}} הסך הן אשכילך | ||
| + | |- | ||
| + | |Write your greater number on a row and write the smaller [number] beneath it on a bottom row. | ||
| + | |style="text-align:right;"|כתוב מיניינך המרובה בטור ותחתיו בטור שפלה כתוב המועט | ||
| + | |- | ||
| + | |Subtract units from units, tens from tens and so on. | ||
| + | |style="text-align:right;"|{{#annot:term|181,1232|59y7}}והוציא{{#annotend:59y7}} אחדי' מן אחדים ועשיריות מן העשיריות וכו‫' | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:46-24|155|KF3P}}Example: to subtract 24 from 46. | ||
| + | :<math>\scriptstyle46-24</math> | ||
| + | |style="text-align:right;"|דומיון להסיר כ"ד מן מ"ו{{#annotend:KF3P}} | ||
| + | |- | ||
| + | | | ||
| + | :Write as follows: | ||
| + | |style="text-align:right;"|כתוב כך | ||
| + | |- | ||
| + | | | ||
| + | :{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |4||6 | ||
| + | |- | ||
| + | |2||4 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| | | | ||
| − | |style="text-align: | + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" |
|- | |- | ||
| | | | ||
| − | |||
| − | |||
{|style="margin-left: auto; margin-right: 0px;" | {|style="margin-left: auto; margin-right: 0px;" | ||
|- | |- | ||
| − | | | + | |ד||ו |
| + | |- | ||
| + | |ב||ד | ||
| + | |} | ||
|- | |- | ||
| − | |||
|} | |} | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|הסר ד' מן ו' וישאר ב' | + | :*Subtract 4 from 6, 2 remains. <math>\scriptstyle{\color{blue}{6-4=2}}</math> |
| + | |style="text-align:right;"|הסר ד' מן ו' וישאר ב‫' | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"|לעולם כדרך זה תלך אחר שתדע שבמעלה האחרונה שהאות גדולה מחבירת' התחתונ' אז לא תחוש בגדול | + | :*Subtract 2 from 4, 2 remains, which is in the second rank. <math>\scriptstyle{\color{blue}{6-4=2}}</math> |
| + | |style="text-align:right;"|{{#annot:term|181,1252|0CKI}}הסר{{#annotend:0CKI}} ב' מן ד' וישאר ב' והיא במעלה שנייה | ||
| + | |- | ||
| + | | | ||
| + | :It is 22. | ||
| + | :<math>\scriptstyle{\color{blue}{46-24=22}}</math> | ||
| + | |style="text-align:right;"|ויהיה ב"ב | ||
| + | |- | ||
| + | |<span style=color:red>[Borrowing one for the preceding rank:]</span> Always follow this way: when you know that the [upper] digit [of the minuend] is greater than its corresponding the bottom digit [of the subtrahend], not considering the measure of the others - always add ten to the upper digit, from which you cannot subtract the [corresponding] bottom [digit]. | ||
| + | |style="text-align:right;"|לעולם כדרך זה תלך אחר שתדע שבמעלה האחרונה שהאות גדולה מחבירת' התחתונ' אז לא תחוש בגדול האחרים צרוף לעולם עשר אל כל אות עליונ' שלא תוכל ליקח ממנו תחתונות | ||
| + | |- | ||
| + | |The beginning [of the procedure] is from the first digit [= the units]. | ||
| + | |style="text-align:right;"|ויהיה התחלתך באות ראשונה | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:2000-1999|155|IREb}} Example: | ||
| + | :<math>\scriptstyle2000-1999</math> | ||
| + | |style="text-align:right;"|דומיון{{#annotend:IREb}} | ||
| + | |- | ||
| + | | | ||
| + | :{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |2||‫0||‫0||‫0 | ||
| + | |- | ||
| + | |1||9||9||9 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
|- | |- | ||
| | | | ||
| − | |||
| − | |||
{|style="margin-left: auto; margin-right: 0px;" | {|style="margin-left: auto; margin-right: 0px;" | ||
|- | |- | ||
| − | + | |ב||‫0||‫0||‫0 | |
| + | |- | ||
| + | |א||ט||ט||ט | ||
| + | |} | ||
|- | |- | ||
| − | |||
|} | |} | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|הסר ט' מי' ונשאר אחד הסר ט' מט' כי דחינו אחד לעניינו וישאר 0' הסר ט' שלישי' מט' וישאר 0' הסר א' מא' וישאר 0' ואעפ"י שיש מאותו אחד נולד לנו כל העשיריות שעשינו כי דחינו הא' אל הט' שלפניה והיא | + | :*Subtract 9 from 10, one remains. <math>\scriptstyle{\color{blue}{10-9=1}}</math> |
| + | |style="text-align:right;"|הסר ט' מי' ונשאר אחד | ||
| + | |- | ||
| + | | | ||
| + | :*Subtract 9 from 9, since we have shifted one for our matter, 0 remains. <math>\scriptstyle{\color{blue}{9-9=0}}</math> | ||
| + | |style="text-align:right;"|הסר ט' מט' כי דחינו אחד לעניינו וישאר 0‫' | ||
| + | |- | ||
| + | | | ||
| + | :*Subtract 9 from the third 9, 0 remains. <math>\scriptstyle{\color{blue}{9-9=0}}</math> | ||
| + | |style="text-align:right;"|הסר ט' שלישי' מט' וישאר 0‫' | ||
| + | |- | ||
| + | | | ||
| + | :*Subtract 1 from 1, 0 remains. <math>\scriptstyle{\color{blue}{1-1=0}}</math> | ||
| + | |style="text-align:right;"|הסר א' מא' וישאר 0‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Although only one [is borrowed], from it all the applied tens are generated: | ||
| + | |style="text-align:right;"|ואעפ"י שיש מאותו אחד נולד לנו כל העשיריות שעשינו | ||
| + | |- | ||
| + | | | ||
| + | ::*For, we have shifted the 1 to the preceding 9, so it is 10, from which 9 is subtracted and 1 remains. | ||
| + | |style="text-align:right;"|כי דחינו הא' אל הט' שלפניה והיא י' לקחת ממנה ט' נותר א‫' | ||
| + | |- | ||
| + | | | ||
| + | ::*We shifted this 1 to the preceding 0, from which 9 is subtracted and 1 remains. | ||
| + | |style="text-align:right;"|הא' הזאת דחינו אל ה0' שלפניה כדי ליקח ממנו ט' וכל מ"י ונשאר א‫' | ||
| + | |- | ||
| + | | | ||
| + | ::*We shifted this 1 to the first 0, so it is 10. Subtract the first 9 from the 10 and 1 remains on 1999, which is one. | ||
| + | :<math>\scriptstyle{\color{blue}{2000-1999=1}}</math> | ||
| + | |style="text-align:right;"|אותה א' דחינו אל ה0' הראשונה ויהיה י' הסר מן הי' ט' הראשונה וישאר א' על ט'ט'ט'א' והוא אחד | ||
| + | |- | ||
| + | |You can understand any number as this one and the other according to this way. | ||
| + | |style="text-align:right;"|ועל דרך זה תוכל להבין כל מניין כזה וזולתו | ||
|- | |- | ||
|} | |} | ||
| Line 254: | Line 486: | ||
== Multiplication == | == Multiplication == | ||
| − | + | |style="text-align:right;"|<big>שער הכפל</big> | |
| + | |- | ||
| + | |To know the multiplication of a number by a number, write one number on the top row and on the bottom row another number. | ||
| + | |style="text-align:right;"|לידע {{#annot:term|156,1230|aYSZ}}כפל{{#annotend:aYSZ}} חשבון על חשבון כתוב מניין אחד בטור העליון ובטור שפל מניין אחר | ||
|- | |- | ||
| + | |Write them like this: write the first digit of the bottom [number] beneath the last digit of the upper [number]. | ||
| + | |style="text-align:right;"|וככה תכתבם כתוב אות ראשון של התחתון תחת אות אחרונה של העליון | ||
| + | |- | ||
| + | |<span style=color:red>The procedure: multiplying and shifting</span> | ||
| | | | ||
| − | |||
|- | |- | ||
| | | | ||
| − | + | :If you multiply the last bottom digit by the last upper digit, write the result above the last bottom digit. | |
| − | |style="text-align:right;"|אם תכפול אות אחרונה שלמטה על אות אחרונה של מעלה | + | |style="text-align:right;"|אם {{#annot:term|185,1230|OCbf}}תכפול{{#annotend:OCbf}} אות אחרונה שלמטה על אות אחרונה של מעלה והעולה כתוב על אות אחרונה של מטה |
|- | |- | ||
| | | | ||
| − | :* | + | :*<span style=color:red>interim product is of the units</span> |
| − | |style="text-align:right;"|אם יעלה הכפל פחות | + | ::If the result is less than 9, write the units above the last bottom digit (and shift the tens one rank forwards). |
| + | |style="text-align:right;"|אם יעלה הכפל פחות מט' כתוב הפרט על אות אחרונה של מטה והכלל תרחיק מעלה ממנו | ||
|- | |- | ||
| | | | ||
| − | :* | + | :*<span style=color:red>interim product is of the tens</span> |
| + | ::If there are no units, only tens, write 0 above the last bottom digit and shift the tens [forwards]. | ||
|style="text-align:right;"|ואם אין פרט רק כלל כתוב 0' על אות אחרונה של מטה והכלל תרחיק וה0' תשי' למעלה | |style="text-align:right;"|ואם אין פרט רק כלל כתוב 0' על אות אחרונה של מטה והכלל תרחיק וה0' תשי' למעלה | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"|ואחריו כפול האות שלפני האות אחרונה שלמטה ג"כ על אות אחרונה שבטור העליון והעולה כתוב אל אותה אות שלמטה שכפלת אות' וכן עשה לכל האותיות כיפלם על אות אחרונה ולאחר שתכפלם כל טור שלמטה על אות אחרונה שלמעלה תשיב אחורני' | + | :Then, multiply also the digit that precedes the last digit in the bottom row by the last digit in the top row and write the product above the bottom digit, by which you have multiplied. |
| + | |style="text-align:right;"|ואחריו כפול האות שלפני האות אחרונה שלמטה ג"כ על אות אחרונה שבטור העליון והעולה כתוב אל אותה אות שלמטה שכפלת אות‫' | ||
| + | |- | ||
| + | | | ||
| + | :Do the same for all the [bottom] digits - multiply them by the last [upper] digit. | ||
| + | |style="text-align:right;"|וכן עשה לכל האותיות כיפלם על אות אחרונה | ||
| + | |- | ||
| + | | | ||
| + | :After you have multiplied all the bottom row by the last upper digit, shift back the digits of the bottom row. | ||
| + | |style="text-align:right;"|ולאחר שתכפלם כל טור שלמטה על אות אחרונה שלמעלה תשיב אחורני' אילו האותיות של טור השפל | ||
| + | |- | ||
| + | | | ||
| + | :Multiply the last bottom digit by the digit that precedes the [last digit] in the top row, then the bottom digit that precedes the last [digit] by the same [upper digit], by which you have multiplied the last [digit], and likewise for all by the same [digit]. | ||
| + | |style="text-align:right;"|וכפול אותי' אחרוני' שלמטה על אות שלפני הראשוני' שכנגד העליון וכן האות שלמטה שלאחר האחרונה על אותה שכפלת האחרונה וכן כולם על אותה | ||
|- | |- | ||
| | | | ||
| − | + | :If there are more than two ranks in the top row, shift back [the bottom row] again as I explained to you. | |
|style="text-align:right;"|ואם יש יותר מב' מעלות בטור עליון תשיב אותה אחורנית עוד כאשר פירשתי לך | |style="text-align:right;"|ואם יש יותר מב' מעלות בטור עליון תשיב אותה אחורנית עוד כאשר פירשתי לך | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|דומיון על כפול כ"ג על כ"ג | + | *{{#annot:23×23|156|FKmC}}Example: multiply 23 by 23. |
| + | :<math>\scriptstyle23\times23</math> | ||
| + | |style="text-align:right;"|דומיון על כפול כ"ג על כ"ג{{#annotend:FKmC}} | ||
| + | |- | ||
| + | |} | ||
| + | :{| | ||
| + | |- | ||
| + | | <span style="color:red>2</span>3||rowspan="2"|<math>\scriptstyle\xrightarrow{{\color{red}{2\times2}}={\color{blue}{4}}}</math>||<span style="color:#0000FF>4</span><span style="color:red>2</span>3||rowspan="2"|<math>\scriptstyle\xrightarrow{{\color{red}{2\times3}}={\color{blue}{6}}}</math>||4<span style="color:#0000FF>6</span><span style="color:red>3</span>||rowspan="2"|<math>\scriptstyle\xrightarrow{\scriptstyle\begin{cases}\scriptstyle{\color{red}{6+\left(3\times2\right)}}={\color{green}{1}}{\color{blue}{2}}\\\scriptstyle{\color{red}{4+}}{\color{green}{1}}={\color{blue}{5}}\end{cases}}</math>||<span style="color:#0000FF>52</span><span style="color:red>3</span>||rowspan="2"|<math>\scriptstyle\xrightarrow{{\color{red}{3\times3}}={\color{blue}{9}}}</math>||52<span style="color:#0000FF>9</span> | ||
| + | |- | ||
| + | |<span style="color:red">2</span>3 ||2<span style="color:red">3</span> || <span style="color:red">2</span>3|| 2<span style="color:red">3</span>|| | ||
| + | |} | ||
| + | |||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | :To know how much is the result, write like this: | ||
| + | |style="text-align:right;"|לדע כמה עולה כתוב כגון זה | ||
| + | |- | ||
| + | | | ||
| + | :{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | | ||2||3 | ||
| + | |- | ||
| + | |2||3|| | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | | ||ב||ג | ||
| + | |- | ||
| + | |ב||ג|| | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | :*Multiply the bottom 2 by the upper 2, it is 4. <math>\scriptstyle{\color{blue}{2\times2=4}}</math> | ||
| + | |style="text-align:right;"|כפול הב' שלמטה על הב' של מעלה ויהי' ד‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Write the 4 in the third rank after the upper 2, like this: | ||
| + | |style="text-align:right;"|אותה הד' כתוב במעלה השלישית אחר ב' העליונה כגון זה | ||
| + | |- | ||
| + | | | ||
| + | :{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |4||2||3 | ||
| + | |- | ||
| + | |2||3|| | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ד||ב||ג | ||
| + | |- | ||
| + | |ב||ג|| | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | :*Multiply also the 3 by 2 that is above it, it is 6. <math>\scriptstyle{\color{blue}{3\times2=6}}</math> | ||
| + | |style="text-align:right;"|כפול הג' ג"כ על הב' שעליה ויהיה ו‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Erase the upper 2 and write the 6 instead, such as: | ||
| + | |style="text-align:right;"|מחק הב' העליונה וכתוב הו' במקומה והדומיון | ||
| + | |- | ||
| + | | | ||
| + | :{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |4||6||3 | ||
| + | |- | ||
| + | |2||3|| | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
|- | |- | ||
| | | | ||
| − | |||
{|style="margin-left: auto; margin-right: 0px;" | {|style="margin-left: auto; margin-right: 0px;" | ||
|- | |- | ||
| − | | | + | |ד||ו||ג |
| + | |- | ||
| + | |ב||ג|| | ||
| + | |} | ||
|- | |- | ||
| − | |||
|} | |} | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*Write the [bottom] 23 beneath the second 3, it is: |
| + | |style="text-align:right;"|כתוב הג"ב האחרונים תחת הג' השנייה ויהיה | ||
| + | |- | ||
| + | | | ||
| + | :{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
{|style="margin-left: auto; margin-right: 0px;" | {|style="margin-left: auto; margin-right: 0px;" | ||
|- | |- | ||
| − | | | + | |4||6||3 |
|- | |- | ||
| − | | | + | | ||2||3 |
|} | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
|- | |- | ||
| | | | ||
| − | |||
{|style="margin-left: auto; margin-right: 0px;" | {|style="margin-left: auto; margin-right: 0px;" | ||
|- | |- | ||
| − | + | |ד||ו||ג | |
|- | |- | ||
| − | | | + | | ||ב||ג |
|} | |} | ||
| − | + | |- | |
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | :*Multiply also the 2 by the 3, it is 12. <math>\scriptstyle{\color{blue}{2\times3{+\color{red}{6}}=12}}</math> | ||
| + | |style="text-align:right;"|כפול הב' על הג' ויהיה י"ב | ||
| + | |- | ||
| + | | | ||
| + | ::Erase the 6 and write the 2 instead. | ||
| + | |style="text-align:right;"|מחק הו' וכתוב ב' במקומה | ||
| + | |- | ||
| + | | | ||
| + | ::Add the 1, which is in the third rank, to the 4, it is 5, such as: | ||
| + | |style="text-align:right;"|והא' שהיא שלישי' במעלה צרוף אותה אל הד' ויהיה ה' הדומיון כך | ||
| + | |- | ||
| + | | | ||
| + | :{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
{|style="margin-left: auto; margin-right: 0px;" | {|style="margin-left: auto; margin-right: 0px;" | ||
|- | |- | ||
| − | | | + | |5||2||3 |
|- | |- | ||
| − | + | | ||2||3 | |
|} | |} | ||
| − | + | |- | |
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
{|style="margin-left: auto; margin-right: 0px;" | {|style="margin-left: auto; margin-right: 0px;" | ||
|- | |- | ||
| − | | | + | |ה||ב||ג |
| + | |- | ||
| + | | ||ב||ג | ||
| + | |} | ||
|- | |- | ||
| − | |||
|} | |} | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|כפול הג' אל הג' ויהיה ט' | + | :*Multiply the 3 by the 3, it is 9. <math>\scriptstyle{\color{blue}{3\times3=9}}</math> |
| + | |style="text-align:right;"|כפול הג' אל הג' ויהיה ט‫' | ||
|- | |- | ||
| | | | ||
| + | ::[Write] it is also above 23, it is [5]29. | ||
| + | :<math>\scriptstyle{\color{blue}{23\times23={\color{red}{5}}29}}</math> | ||
| + | |style="text-align:right;"|זה ג"כ על ג"ב הוא ט'ב'א‫' | ||
| + | |- | ||
| + | |According to this way you can know the multiplication of everything. | ||
|style="text-align:right;"|ועל דרך זה תוכל לידע כל הכפל דבר לכפול דבר | |style="text-align:right;"|ועל דרך זה תוכל לידע כל הכפל דבר לכפול דבר | ||
|- | |- | ||
| − | | | + | |To multiply three digits by three digits: |
| − | |style="text-align:right;"|לכפול ג' אותיות על ג' אותיות כפול התחתון על השניות במעלה תשיב אחורנית וכפול אותם ג"כ במעלה ראשונה וכפול אותם על אות התחתונה שתכפול ומה | + | |style="text-align:right;"|לכפול ג' אותיות על ג' אותיות |
| + | |- | ||
| + | | | ||
| + | :Multiply the bottom [digits] by the second rank, then shift back and multiply them also by the first rank. Multiply it by the bottom digit, then erase what is above it. | ||
| + | |style="text-align:right;"|כפול התחתון על השניות במעלה תשיב אחורנית וכפול אותם ג"כ במעלה ראשונה וכפול אותם על אות התחתונה שתכפול ומה שלמעלה תמחוק | ||
|- | |- | ||
|} | |} | ||
| Line 338: | Line 733: | ||
== Division == | == Division == | ||
| − | + | |style="text-align:right;"|<big>שער ה{{#annot:term|157,1223|Vnia}}חלוק{{#annotend:Vnia}}</big> | |
| + | |- | ||
| + | |If a person asks you: how many times the small number is in the greater number? | ||
| + | |style="text-align:right;"|אם ישאלך אדם כמה פעמים סך הקטון בסך הגדול | ||
| + | |- | ||
| + | |Write the greater number in one row according to its ranks, then write the smaller number beneath it in a bottom row. Write the last bottom digit beneath the last upper digit. | ||
| + | |style="text-align:right;"|כתוב החשבון הגדול בטור אחד לפי מעלות ותחתיו כתוב בטור שפל סך הקטון ואות אחרונה של השפל כתוב אותה תחת אות אחרונה של העליון | ||
| + | |- | ||
| + | |See how many times the bottom [number] is in the upper [number] and write the result in a third row beneath the first digit of the second row. | ||
| + | |style="text-align:right;"|וראה כמה פעמים התחתון בעליון והעולה תכתוב אותה בטור שלישי תחת אות ראשונה שבטור שניה | ||
| + | |- | ||
| + | |As [the number of] times that you take the last [bottom] digit from the last [upper digit] so is [the number of] times that you take the other [bottom] digits from the upper number. | ||
| + | |style="text-align:right;"|וכל כך פעמים שתקח אות אחרונה מן האחרונה כל פעמים קח האותיו' האחרות מן הסך העליון | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *{{#annot:654÷70|157|074J}}Here is an example: we want to know how many times 70 there are in 654? |
| + | :<math>\scriptstyle654\div70</math> | ||
| + | |style="text-align:right;"|והא לך דומיון ביקשנו לדע כמה פעמים ע' בתוך תרנ"ד{{#annotend:074J}} | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*Write 654 in this row. |
| + | |style="text-align:right;"|תכתוב תרנ"ד בטור זה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*Then, write 70 in the bottom row, like this: |
| + | |style="text-align:right;"|ואחר כתוב ע' בטור שפל כזה | ||
| + | |- | ||
| + | | | ||
| + | :{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |6||5||4 | ||
| + | |- | ||
| + | | ||7||0 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
|- | |- | ||
| | | | ||
| − | |||
{|style="margin-left: auto; margin-right: 0px;" | {|style="margin-left: auto; margin-right: 0px;" | ||
|- | |- | ||
| − | + | |ו||ה||ד | |
|- | |- | ||
| − | + | | ||ז||‫0 | |
|} | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::If we would write the last 7 beneath the 6, which is also last [digit], we would not find 7 in it. | ||
| + | |style="text-align:right;"|אומנם אם נכתב ז' אחרונה תחת הו' שהיא גם כן אחרונה לא נוכל למצוא ז' בו | ||
| + | |- | ||
| + | | | ||
| + | :*Therefore, we have to write the 7 corresponding to the 5 of 654. | ||
| + | |style="text-align:right;"|לכן אנו צריכין לכתוב הז' כנגד הה' של דה"ו ‫[...] | ||
| + | |- | ||
| + | | | ||
| + | :*Say: how many times 7 there are in 65? | ||
| + | |style="text-align:right;"|אמור כמה פעמים ז' בס"ה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::We answer: 9 times. |
| + | ::<math>\scriptstyle{\color{blue}{65=\left(7\times9\right){\color{red}{+r}}}}</math> | ||
| + | |style="text-align:right;"|נאמר ט' פעמים אות' ט‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|דומיון אחר בקשנו לידע כמה פעמים י"ט בתוך שמנים ושש וחמש אלפים | + | :*Write this 9 in a third row beneath the 0. |
| + | |style="text-align:right;"|כתוב בטור שלישי' תחת ה0‫' | ||
| + | |- | ||
| + | | | ||
| + | ::2 remains instead of the 5 of 654, and with the 4 of 654, which we did not divide yet, they are 24. | ||
| + | |style="text-align:right;"|וישאר ב' במקו' ה' של דה"ו והד' שלפני דה"ו בב' שלא חלקנו עדיין כ"ד | ||
| + | |- | ||
| + | | | ||
| + | ::Hence we find that 70 is in 654 9 times and 24 remains. | ||
| + | :<math>\scriptstyle{\color{blue}{654=\left(70\times9\right)+24}}</math> | ||
| + | |style="text-align:right;"|א"כ מצאנו ע' בתוך תרנ"ד ט' פעמים וישאר כ"ד | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:5086÷19|157|o1gb}}Another example: we want to know: how many times 19 there are in 5086? | ||
| + | :<math>\scriptstyle5086\div19</math> | ||
| + | |style="text-align:right;"|דומיון אחר בקשנו לידע כמה פעמים י"ט בתוך שמנים ושש וחמש אלפים{{#annotend:o1gb}} | ||
| + | |- | ||
| + | |} | ||
| + | :{| | ||
| + | |- | ||
| + | |<span style="color:red>50</span>86||rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{red}{50-\left({\color{blue}{2}}\times19\right)}}={\color{blue}{12}}}</math>||<span style="color:#0000FF>12</span><span style="color:red>8</span>6||rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{red}{128-\left({\color{blue}{6}}\times19\right)}}={\color{blue}{14}}}</math>||<span style="color:#0000FF>14</span><span style="color:red>6</span>||rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{red}{146-\left({\color{blue}{7}}\times19\right)}}={\color{blue}{13}}}</math>|| <span style="color:#0000FF>13</span> | ||
| + | |- | ||
| + | |<span style="color:red">19</span>  ||<span style="color:red">19</span>  ||<span style="color:red">19</span> ||19  | ||
| + | |- | ||
| + | | || <span style="color:#0000FF">2</span> ||2<span style="color:#0000FF">6</span> ||26<span style="color:#0000FF">7</span> | ||
| + | |} | ||
| + | |||
| + | {| | ||
|- | |- | ||
| | | | ||
| + | :*Write the greater number according to agorima, then write the 19 beneath is, like this: | ||
|style="text-align:right;"|כתוב מניין הגדול על פי אגורימא וכתוב תחתיו הי"ט כזה | |style="text-align:right;"|כתוב מניין הגדול על פי אגורימא וכתוב תחתיו הי"ט כזה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :{|class="wikitable" style="color:blue; text-align:center;" |
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |5||0||8||6 | ||
| + | |- | ||
| + | |1||9|| || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|מצאת י"ט בתוך מעלה אותה ב' כתוב תחת הט' ויהיה דמיונ' כך | + | {|style="margin-left: auto; margin-right: 0px;" |
| + | |- | ||
| + | |ה||0||ח||ו | ||
| + | |- | ||
| + | |א||ט|| || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | :*Say: how many times the bottom 1 is in the upper 5? | ||
| + | |style="text-align:right;"|אמור כמה פעמים א' שפלה בתוך ה' של מעלה | ||
| + | |- | ||
| + | | | ||
| + | ::*If you would take 5 times, from what would you take 5 times the 9? | ||
| + | |style="text-align:right;"|אם תיקח ה' פעמים ואז ממה תקח הט' ה' פעמים | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ואם ד' פעמים ותאמר א' שהיא עשר במקום ה0' שלפניה לא תוכל ליקח ט' ד' פעמים מעשר‫' | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ואם תקח א' ג' פעמים וישאר ב' במקום הב' שהם עשרים במקום ה0' הן לא תוכל ליקח הט' ג' פעמי' מעשרים | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|אכן קח הא' ב' פעמים וישאר במקום הה' ג' קח ב' מהג' והשב אחורנית מקום ה0' ויהיה עשרים וקח ט' ב' פעמים מעשרים וישאר ב' במקום ה0' וא' במקום הה' כתוב ב' שכל סך פעמ' מצאת י"ט בתוך מעלה אותה ב' כתוב תחת הט' ויהיה דמיונ' כך | ||
| + | |- | ||
| + | | | ||
| + | :{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |1||2||8||6 | ||
| + | |- | ||
| + | |1||9|| || | ||
| + | |- | ||
| + | | ||2|| || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |א||ב||ח||ו | ||
| + | |- | ||
| + | |א||ט|| || | ||
| + | |- | ||
| + | | ||ב|| || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
|- | |- | ||
| | | | ||
| Line 377: | Line 911: | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|ואמור כמה פעמים א' בב"א | + | :{|class="wikitable" style="color:blue; text-align:center;" |
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |1||2||8||6 | ||
| + | |- | ||
| + | | ||1||9|| | ||
| + | |- | ||
| + | | ||2|| || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |א||ב||ח||ו | ||
| + | |- | ||
| + | | ||א||ט|| | ||
| + | |- | ||
| + | | ||ב|| || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ואמור כמה פעמים א' בב"א וקח אותה כל כך מעט כדי שתוכל ליקח הט' גם כל כך ז' פעמים לא תוכל ליקח הט' מן הנשאר ז' פעמים כי מן הב"א ישאר ו"ח שעל הט' זהו ח"ה לא תשיג ליקח ממנו ז' פעמים ט' כי הוא עולה יותר אכן קח מן מ"ו פעמים מן הי"ב שעליה וישאר ו' במקו' הב' כזה | ||
| + | |- | ||
| + | | | ||
| + | :{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |6||8||6 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ו||ח||ו | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
|- | |- | ||
| | | | ||
|style="text-align:right;"|השב הט' אחורנית תחת הו' העליונה קח ו' פעמי' ט' מח"ו וישאר במקום הח' ד' ובמקום הו' א' כזה | |style="text-align:right;"|השב הט' אחורנית תחת הו' העליונה קח ו' פעמי' ט' מח"ו וישאר במקום הח' ד' ובמקום הו' א' כזה | ||
| + | |- | ||
| + | | | ||
| + | :{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |1||4||6 | ||
| + | |- | ||
| + | |1||9|| | ||
| + | |- | ||
| + | |2|| || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |א||ד||ו | ||
| + | |- | ||
| + | |א||ט|| | ||
| + | |- | ||
| + | |ב|| || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
|- | |- | ||
| | | | ||
| Line 386: | Line 999: | ||
|- | |- | ||
| | | | ||
| − | |style="text-align: | + | :{|class="wikitable" style="color:blue; text-align:center;" |
|- | |- | ||
| | | | ||
| − | |style=" | + | {|style="margin-left: auto; margin-right: 0px;" |
| + | |- | ||
| + | |1||4||6 | ||
| + | |- | ||
| + | |1||9|| | ||
| + | |- | ||
| + | |2||6|| | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|וזה יורה לך | + | {|style="margin-left: auto; margin-right: 0px;" |
| + | |- | ||
| + | |א||ד||ו | ||
| + | |- | ||
| + | |א||ט|| | ||
| + | |- | ||
| + | |ב||ו|| | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|השב הט' אחורנית תחת הו' עליונה כזה | ||
| + | |- | ||
| + | | | ||
| + | :{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |1||4||6 | ||
| + | |- | ||
| + | | ||1||9 | ||
| + | |- | ||
| + | |2||6|| | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |א||ד||ו | ||
| + | |- | ||
| + | | ||א||ט | ||
| + | |- | ||
| + | |ב||ו|| | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|קח א' שפלה מד"א ז"פ וישאר במקום ז' במקום הד' ויהיה הדומיון כזה | ||
| + | |- | ||
| + | | | ||
| + | :{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | | ||7||6 | ||
| + | |- | ||
| + | | ||1||9 | ||
| + | |- | ||
| + | |2||6|| | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | | ||ז||ו | ||
| + | |- | ||
| + | | ||א||ט | ||
| + | |- | ||
| + | |ב||ו|| | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|קח הט' גם כן ז' פעמים מו"ז וישאר ג' במקום הו' וא' במקום הז' יכתוב הז' שמצאת' תחת הט' ויהיה הדומיון כך | ||
| + | |- | ||
| + | | | ||
| + | :{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | | ||1||3 | ||
| + | |- | ||
| + | | ||1||9 | ||
| + | |- | ||
| + | |2||6||7 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | | ||א||ג | ||
| + | |- | ||
| + | | ||א||ט | ||
| + | |- | ||
| + | |ב||ו||ז | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|וזה יורה לך שמצאת ט"א בתוך וח0"ה במעלה השלישית שהם מאות ו' במעלה השנייה שהם עשיריו' וז' במעלה ראשונה שהם אחדים וישארו ג"א שלא באו לחלק א"כ הן כמניין הגדול וז' פעמי' וג"א חלקים בשלימות ועל דרך זה תחלוק | ||
|- | |- | ||
|} | |} | ||
| Line 401: | Line 1,133: | ||
== Proportions == | == Proportions == | ||
| − | !style="text-align:right;"|שער | + | !style="text-align:right;"|שער ה{{#annot:term|564,1367|I9RW}}ערוך{{#annotend:I9RW}} |
|- | |- | ||
| | | | ||
| Line 407: | Line 1,139: | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|כאשר ישאל השואל הנה ידוע לך שי"א תרנגולים נמכרים | + | *{{#annot:roosters|629|DWbY}}When one asks: you know that 11 roosters are sold for 21 pešiṭim. Now, you ask: what is the price of 8 roosters? |
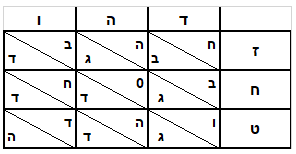
| + | :<math>\scriptstyle\frac{11}{21}=\frac{8}{X}</math> | ||
| + | |style="text-align:right;"|כאשר ישאל השואל הנה ידוע לך שי"א תרנגולים נמכרים בכ"א פ' ועתה תשאל היאך ערך ח' תרנגולים{{#annotend:DWbY}} | ||
|- | |- | ||
| | | | ||
| Line 413: | Line 1,147: | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|ואמור | + | :{|class="wikitable" style="color:blue; text-align:center;" |
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |21||11 | ||
| + | |- | ||
| + | | ||8 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |אב||אא | ||
| + | |- | ||
| + | | ||ח | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ואמור כ{{#annot:term|482,1280|TXuZ}}ערך{{#annotend:TXuZ}} א"א על א"ב כן ערך א' על הנסתר | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|כפול ח' על הנסתר א"ב ויעלה חו"א | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|חלקיהא בא"א ותמצא כ"ו ט"ו פעמים א"א בתוך חו"א וישאר ג' שלא תוכל לחלוק | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|א"כ ח' תרנגולים ימכרו עבור ט"ו וג' {{#annot:term|15,1242|yiqq}}שברים{{#annotend:yiqq}} מן א"א כ{{#annot:term|20,1268|68kh}}שלמין{{#annotend:68kh}} | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|דומיון הנה קניתי י"ב אמות בגד בכ"ח דינ' וביקשת לידע כמה ערך של ז' אמות | + | *{{#annot:cloth|629|TKpg}}Example: I bought 12 cubits of cloth for 28 dinar. You wish to know: what is the price of 7 cubits? |
| + | :<math>\scriptstyle\frac{12}{28}=\frac{7}{X}</math> | ||
| + | |style="text-align:right;"|דומיון הנה קניתי י"ב אמות בגד בכ"ח דינ' וביקשת לידע כמה ערך של ז' אמות{{#annotend:TKpg}} | ||
|- | |- | ||
| | | | ||
| Line 422: | Line 1,192: | ||
|- | |- | ||
| | | | ||
| − | |style="text-align: | + | :{|class="wikitable" style="color:blue; text-align:center;" |
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |28||12 | ||
| + | |- | ||
| + | | ||8 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |חב||בא | ||
| + | |- | ||
| + | | ||ח | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ויאמר כערך י"ב על ט"ו כן ערך הנסתר אל ז' |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וככה תעשה כפל ח"ב בז' ויעלה וט"א |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|ואמר כערך ז"א אל א"ד כך ערך 0"ג אל הנסתר כפול ז"א על א"ד והם זט"ו חלקם | + | |style="text-align:right;"|חלקם בב"א ויעלה ו"א וד' מי"ב ב{{#annot:term|20,1268|zWcd}}שלימים{{#annotend:zWcd}} |
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|א"כ ערך הז' אמות י"ז דינר וד' מי"ב בשלימי‫' | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:one worker|612|9ct6}}Example: you hired a worker for 30 days for 41 dinar, but he worked with you only 17 days. You wish to know: how much should his payment for these days be? | ||
| + | :<math>\scriptstyle\frac{30}{41}=\frac{17}{X}</math> | ||
| + | |style="text-align:right;"|דומיון הנה שכרתה שכיר ל' ימים במ"א דינר ולא עבד אתך אלא י"ז ימים ובקשת לידע כמה שכרו לאותו הימים{{#annotend:9ct6}} | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|כתוב ל' והם הדמים אשר היה לו לעבוד בטור העליון ואחריו מ"א באותו הטור והם דמי השכיר אם עבד עמך כל הימים וכתוב בטור שנייה תחתיו הל' י"ז והם אשר עבד | ||
| + | |- | ||
| + | |Written according to agorima as follows | ||
| + | |style="text-align:right;"|יכתוב אותם על פי אגורימא כזה | ||
| + | |- | ||
| + | | | ||
| + | :{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |41||30 | ||
| + | |- | ||
| + | | ||17 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |אד||‫0ג | ||
| + | |- | ||
| + | | ||זא | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ואמר כערך ז"א אל א"ד כך ערך 0"ג אל הנסתר | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|כפול ז"א על א"ד והם זט"ו | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|{{#annot:term|784,1259|k7XL}}חלקם ב{{#annotend:k7XL}}0"ג ויעלה ג"ב וז' חלקים מ0"ג בשלמי' | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|א"כ אתה חייב לו מן העבודה אשר עבד אתך הי"ז ימים כ"ג דינר וז' פרוטות מל' בדינר | ||
|- | |- | ||
| | | | ||
|style="text-align:right;"|סליק שער הערוך | |style="text-align:right;"|סליק שער הערוך | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | == Gelosia == | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|פי' זה הלוח | ||
| + | |- | ||
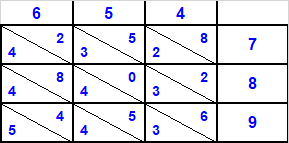
| + | |[[File:Agris - Gelosia - 2.png|thumb|300px|left]] | ||
| + | |[[File:Agris - Gelosia - 1.png|thumb|300px]] | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|עשה עמו כפל בלי מחק כאשר רמזתי תקן | ||
| + | |- | ||
| + | | | ||
| + | *<math>\scriptstyle654\times987</math> | ||
| + | |style="text-align:right;"|ותאמר אם תרצה לידע כמה עולה ד'ה'ו' אז תכתוב כמו שכתבתי ז'ח'ט' | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle7\times4=28</math> | ||
| + | |style="text-align:right;"|ותאמר ז' פעמי' ד' הרי כ"ח | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle7\times5=35</math> | ||
| + | |style="text-align:right;"|ז' פעמי' ה' הרי ל"ה | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle7\times6=42</math> | ||
| + | |style="text-align:right;"|ז' פעמים ו' הרי מ"ב | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|וכן תעשה לכולם | ||
| + | |- | ||
| + | | | ||
| + | :the result: <math>\scriptstyle{\color{blue}{654\times987=645498}}</math> | ||
| + | |style="text-align:right;"|ח'ט'ד'ה'ד'ו' זה עולה | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|פי' זה הלוח לידע כמה הוה ב"פ ב' ג"פ ג' ד"פ ד' ה"פ ה' וכן כולם | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|סליק ספר צאיפור | ||
|- | |- | ||
|} | |} | ||
| + | == Appendix: Bibliography == | ||
| + | '''''Anonymous'''''<br> | ||
| + | '''''Sefer Agris / Agrima / Sefer Ṣaifur / Ṣifur'''''<br> | ||
| + | |||
| + | |||
| + | '''Manuscripts:'''<br> | ||
| + | :1) Darmstadt, Hessisches Landes-und Hochschulbibliothek Cod. Or. 25/39 (IMHM: f 12695), ff. 71v-73r (14th century)<br> | ||
| + | :[http://tudigit.ulb.tu-darmstadt.de/show/cod-or-25/0142?sid=f24f87d226d386c8a0ea3d057e712dc5 Or. 25/39] | ||
| + | :2) Oxford, Bodleian Library MS Opp. 757/17 (IMHM: f 20981), ff. 322v-326v (cat. Neub. 2289,17); (16th century) | ||
| + | :3) Warszaw, Żydowski Instytut Historyczny 288/2 (IMHM: f 12013), ff. 6r-22r (15th century) | ||
| − | = | + | <span style=color:blue>The transcript is based mainly on manuscript Darmstadt</span> |
| − | + | '''Bibliography:'''<br> | |
| − | + | *Steinschneider, Moritz. 1906. Mathematik bei den Juden, Band II: 1551-1840. Monatsschrift für die Geschichte und Wissenschaft des Judenthums 50, p. 213. repr.: ed. Gad Freudenthal, Hildesheim, Zürich, New York: Olms, 2014, p. 136. | |
| − | |||
| − | |||
Latest revision as of 15:17, 8 July 2022
| אתחיל לכתוב ספר חשבונות | |
| שקורין צאיפור | |
| בשם הדר במעונות | |
| Sefer Agris was prepared according to the script [= perhaps an indication that the work relies on another book] | ספר אגריס אשר נעשה על פי הכתב |
| ויש בו שערים אשר בהכנתם תוחלת רב | |
| The treatise apparently consisted of nine chapters | ומיניינם אשר החילותי לפרשם הם ט' |
| ושאריתם לא מצאתי מפרש |
Contents[hide]Introduction: The Positional Decimal System |
|||||||||||
| The numerals | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| The nine numerals - the nine first Hebrew letters | ומהנה אתחיל ואומר האותיות היוצאות במילת אגריס לפועל הם ט' כמספרם ואילו הם | ||||||||||
| 1 2 3 4 5 6 7 8 9 |
| ||||||||||
| Every number is written with these nine numerals | ובהם כתוב כל מספר | ||||||||||
| The written ranks [= decimal places] | |||||||||||
| Units | ודע כל מניין שתחפוץ לכתוב מא' עד ט' | ||||||||||
| written with one letter only | תוכל לכתוב באות אחת כגון אחת א' או שתים ב' כו' עד ט' | ||||||||||
| Ten cannot be indicated by the nine numerals | אך עשרה לא תמצא באותיות האילו הנרמזים אך תכתוב 0"א פי' עשרה עמוד גלגל ואחר תכתוב הא' | ||||||||||
| Zero: 10 - the zero indicates that 1 is in the second rank; 1 without a zero is one | והגלגל יורה שהא' במעלה שנייה והיא עשרה והא' בלא גלגל אחד | ||||||||||
| Tens | וכן כל האותיות עד ט' | ||||||||||
|
ואולם כשיש גלגל לפני האות מורה על העשיריות | ||||||||||
|
וכדרך זה תכתוב אם דעתך לכתוב עשרים תכתוב 0"ב | ||||||||||
|
שלשים 0"ג | ||||||||||
|
ארבעים 0"ד | ||||||||||
| ועל דרך זה תוכל להבין כל אות שהיא במעלה שנייה שהיא עשירית | |||||||||||
|
וכדרך זה תכתוב עד תשעים והוא 0"ט | ||||||||||
| Hundreds | |||||||||||
|
אך מאה היאך תרצה לכתוב תכתוב א' במעלה השלישית כזה 00"א | ||||||||||
|
ב' מאות כזה 00"ב | ||||||||||
|
ג' מאות 00"ג | ||||||||||
|
ד' מאות 00"ד | ||||||||||
|
וכן תעשה עד ט' מאות כזה 00"ט | ||||||||||
| Thousands | |||||||||||
|
רק אלף היאך תרצה לכתוב תכתוב במעלה רביעית כזה 000"א | ||||||||||
|
אלפים כזה 000"ב | ||||||||||
|
ג' אלפים כזה 000"ג | ||||||||||
|
ד' אלפים 000"ד | ||||||||||
|
וכו' עד ט' אלפים כזה 000"ט | ||||||||||
| Tens of thousands | |||||||||||
|
ועשרת אלפים תכתוב כזה 0000"א והא' עומד במעלה החמישית | ||||||||||
|
עשרים אלפים 0000"ב | ||||||||||
|
שלשים אלפים 0000"ג | ||||||||||
|
ארבעים אלפים כזה 0000"ד | ||||||||||
|
וכו' עד תשעים אלפים כזה 0000"ט | ||||||||||
| Hundreds of thousands | |||||||||||
|
מאה אלפי' תכתוב הא' במעלה שישית כזה 00000"א וכו' | ||||||||||
| Every rank is ten times the preceding rank | ועתה תבין ותדע שהא' במעלה ראשונה עשיר' היא שהיא במעלה השנייה והשנייה עשרי' השלישי' והשלישי' עשירי' הרביעי' וכן לעולם כל אשר יתרבו המעלות כן יתרבו המניין ותבין בו כי הוא דרך אמת | ||||||||||
| ומכאן אפרש לך שער המועל סכום לסכום |
Addition |
שער החיבור | ||||||||||||||
| To let you know how to sum two numbers together. | להודיעך איך תתחבר שני חשבונות יחד | ||||||||||||||
| Write one number in a line and beneath is the second number: units corresponding to units and tens corresponding to tens, meaning write the digit [lit. letter] that is in the first rank beneath the digit that is also in the first rank, then the second beneath the second in rank and so on. | תכתוב חשבון אחד בשורה ותחתיו חשבון השני פרט נגד פרט וכלל נגד כלל פי' האות שהיא במעלה הראשונה כתוב האות תחת אות שהיא ג"כ למעלה ראשונה והשנייה תחת השנייה במעלה וכן כולם | ||||||||||||||
|
דומיון רצונך לחבר ד' וארבעים לה' וחמשים | ||||||||||||||
|
תכתוב כך | ||||||||||||||
|
| ||||||||||||||
|
חבר ד' עם ה' שבתחתון והיא ט' | ||||||||||||||
|
חביר ד' שנייה ה' שנייה והוא ט' | ||||||||||||||
|
א"כ מצאנו צירופם ט"ט והם תשעה ותשעים | ||||||||||||||
| Sum all the digits according to this way. | ובזה הדרך חבר כל האותיות | ||||||||||||||
|
דומיון אחר | ||||||||||||||
|
| ||||||||||||||
|
חבר ט' על א' שבתחתה והיא א"א עשרה | ||||||||||||||
|
כתוב 0' ישאר לך א' והיא א' שנייה במעלה | ||||||||||||||
|
צרוף אותה במעלה לא' השנייה גם כן במעלה ויהיה ב' צרוף הב' על הט' במעלה שנייה ויהיה א"א | ||||||||||||||
|
ובתחילה 0' ויהיה 0'א'א' | ||||||||||||||
|
דומיון אחר | ||||||||||||||
|
| ||||||||||||||
|
חבר ב' על ח' זה עשרה | ||||||||||||||
|
כתוב 0' | ||||||||||||||
|
והא' הנותרת חבר עם הו' ויהיה ז' חברה על הד' ויהיה א"א | ||||||||||||||
|
כתוב א' במעלה שנייה | ||||||||||||||
|
והא' השלישי' במעלה חברה על הט' ועל הא' זהו א"א | ||||||||||||||
|
אז הוא 0אא"א |
Subtraction |
שער החסור | ||||||||||||||||||
| If you know a number and you wish to subtract [from it] a certain amount and know what remains from the first number after subtracting the amount from it, I will teach you this: | אם ידעת חשבון ורצונך לחסר מי"ט סך אחד וחפצתה לידע מה יסיר מן החשבון הראשון אחר סלוק הסך הן אשכילך | ||||||||||||||||||
| Write your greater number on a row and write the smaller [number] beneath it on a bottom row. | כתוב מיניינך המרובה בטור ותחתיו בטור שפלה כתוב המועט | ||||||||||||||||||
| Subtract units from units, tens from tens and so on. | והוציא אחדי' מן אחדים ועשיריות מן העשיריות וכו' | ||||||||||||||||||
|
דומיון להסיר כ"ד מן מ"ו | ||||||||||||||||||
|
כתוב כך | ||||||||||||||||||
|
| ||||||||||||||||||
|
הסר ד' מן ו' וישאר ב' | ||||||||||||||||||
|
הסר ב' מן ד' וישאר ב' והיא במעלה שנייה | ||||||||||||||||||
|
ויהיה ב"ב | ||||||||||||||||||
| [Borrowing one for the preceding rank:] Always follow this way: when you know that the [upper] digit [of the minuend] is greater than its corresponding the bottom digit [of the subtrahend], not considering the measure of the others - always add ten to the upper digit, from which you cannot subtract the [corresponding] bottom [digit]. | לעולם כדרך זה תלך אחר שתדע שבמעלה האחרונה שהאות גדולה מחבירת' התחתונ' אז לא תחוש בגדול האחרים צרוף לעולם עשר אל כל אות עליונ' שלא תוכל ליקח ממנו תחתונות | ||||||||||||||||||
| The beginning [of the procedure] is from the first digit [= the units]. | ויהיה התחלתך באות ראשונה | ||||||||||||||||||
|
דומיון | ||||||||||||||||||
|
| ||||||||||||||||||
|
הסר ט' מי' ונשאר אחד | ||||||||||||||||||
|
הסר ט' מט' כי דחינו אחד לעניינו וישאר 0' | ||||||||||||||||||
|
הסר ט' שלישי' מט' וישאר 0' | ||||||||||||||||||
|
הסר א' מא' וישאר 0' | ||||||||||||||||||
|
ואעפ"י שיש מאותו אחד נולד לנו כל העשיריות שעשינו | ||||||||||||||||||
|
כי דחינו הא' אל הט' שלפניה והיא י' לקחת ממנה ט' נותר א' | ||||||||||||||||||
|
הא' הזאת דחינו אל ה0' שלפניה כדי ליקח ממנו ט' וכל מ"י ונשאר א' | ||||||||||||||||||
|
אותה א' דחינו אל ה0' הראשונה ויהיה י' הסר מן הי' ט' הראשונה וישאר א' על ט'ט'ט'א' והוא אחד | ||||||||||||||||||
| You can understand any number as this one and the other according to this way. | ועל דרך זה תוכל להבין כל מניין כזה וזולתו |
Multiplication |
שער הכפל |
| To know the multiplication of a number by a number, write one number on the top row and on the bottom row another number. | לידע כפל חשבון על חשבון כתוב מניין אחד בטור העליון ובטור שפל מניין אחר |
| Write them like this: write the first digit of the bottom [number] beneath the last digit of the upper [number]. | וככה תכתבם כתוב אות ראשון של התחתון תחת אות אחרונה של העליון |
| The procedure: multiplying and shifting | |
|
אם תכפול אות אחרונה שלמטה על אות אחרונה של מעלה והעולה כתוב על אות אחרונה של מטה |
|
אם יעלה הכפל פחות מט' כתוב הפרט על אות אחרונה של מטה והכלל תרחיק מעלה ממנו |
|
ואם אין פרט רק כלל כתוב 0' על אות אחרונה של מטה והכלל תרחיק וה0' תשי' למעלה |
|
ואחריו כפול האות שלפני האות אחרונה שלמטה ג"כ על אות אחרונה שבטור העליון והעולה כתוב אל אותה אות שלמטה שכפלת אות' |
|
וכן עשה לכל האותיות כיפלם על אות אחרונה |
|
ולאחר שתכפלם כל טור שלמטה על אות אחרונה שלמעלה תשיב אחורני' אילו האותיות של טור השפל |
|
וכפול אותי' אחרוני' שלמטה על אות שלפני הראשוני' שכנגד העליון וכן האות שלמטה שלאחר האחרונה על אותה שכפלת האחרונה וכן כולם על אותה |
|
ואם יש יותר מב' מעלות בטור עליון תשיב אותה אחורנית עוד כאשר פירשתי לך |
|
דומיון על כפול כ"ג על כ"ג |
23 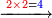
423 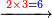
463 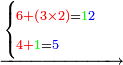
523 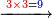
529 23 23 23 23
|
לדע כמה עולה כתוב כגון זה | ||||||||||||||
|
| ||||||||||||||
|
כפול הב' שלמטה על הב' של מעלה ויהי' ד' | ||||||||||||||
|
אותה הד' כתוב במעלה השלישית אחר ב' העליונה כגון זה | ||||||||||||||
|
| ||||||||||||||
|
כפול הג' ג"כ על הב' שעליה ויהיה ו' | ||||||||||||||
|
מחק הב' העליונה וכתוב הו' במקומה והדומיון | ||||||||||||||
|
| ||||||||||||||
|
כתוב הג"ב האחרונים תחת הג' השנייה ויהיה | ||||||||||||||
|
| ||||||||||||||
|
כפול הב' על הג' ויהיה י"ב | ||||||||||||||
|
מחק הו' וכתוב ב' במקומה | ||||||||||||||
|
והא' שהיא שלישי' במעלה צרוף אותה אל הד' ויהיה ה' הדומיון כך | ||||||||||||||
|
| ||||||||||||||
|
כפול הג' אל הג' ויהיה ט' | ||||||||||||||
|
זה ג"כ על ג"ב הוא ט'ב'א' | ||||||||||||||
| According to this way you can know the multiplication of everything. | ועל דרך זה תוכל לידע כל הכפל דבר לכפול דבר | ||||||||||||||
| To multiply three digits by three digits: | לכפול ג' אותיות על ג' אותיות | ||||||||||||||
|
כפול התחתון על השניות במעלה תשיב אחורנית וכפול אותם ג"כ במעלה ראשונה וכפול אותם על אות התחתונה שתכפול ומה שלמעלה תמחוק |
Division |
שער החלוק | ||||||||||||||
| If a person asks you: how many times the small number is in the greater number? | אם ישאלך אדם כמה פעמים סך הקטון בסך הגדול | ||||||||||||||
| Write the greater number in one row according to its ranks, then write the smaller number beneath it in a bottom row. Write the last bottom digit beneath the last upper digit. | כתוב החשבון הגדול בטור אחד לפי מעלות ותחתיו כתוב בטור שפל סך הקטון ואות אחרונה של השפל כתוב אותה תחת אות אחרונה של העליון | ||||||||||||||
| See how many times the bottom [number] is in the upper [number] and write the result in a third row beneath the first digit of the second row. | וראה כמה פעמים התחתון בעליון והעולה תכתוב אותה בטור שלישי תחת אות ראשונה שבטור שניה | ||||||||||||||
| As [the number of] times that you take the last [bottom] digit from the last [upper digit] so is [the number of] times that you take the other [bottom] digits from the upper number. | וכל כך פעמים שתקח אות אחרונה מן האחרונה כל פעמים קח האותיו' האחרות מן הסך העליון | ||||||||||||||
|
והא לך דומיון ביקשנו לדע כמה פעמים ע' בתוך תרנ"ד | ||||||||||||||
|
תכתוב תרנ"ד בטור זה | ||||||||||||||
|
ואחר כתוב ע' בטור שפל כזה | ||||||||||||||
|
| ||||||||||||||
|
אומנם אם נכתב ז' אחרונה תחת הו' שהיא גם כן אחרונה לא נוכל למצוא ז' בו | ||||||||||||||
|
לכן אנו צריכין לכתוב הז' כנגד הה' של דה"ו [...] | ||||||||||||||
|
אמור כמה פעמים ז' בס"ה | ||||||||||||||
|
נאמר ט' פעמים אות' ט' | ||||||||||||||
|
כתוב בטור שלישי' תחת ה0' | ||||||||||||||
|
וישאר ב' במקו' ה' של דה"ו והד' שלפני דה"ו בב' שלא חלקנו עדיין כ"ד | ||||||||||||||
|
א"כ מצאנו ע' בתוך תרנ"ד ט' פעמים וישאר כ"ד | ||||||||||||||
|
דומיון אחר בקשנו לידע כמה פעמים י"ט בתוך שמנים ושש וחמש אלפים |
5086 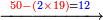
1286 
146 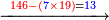
13 19 19 19 19 2 26 267
|
כתוב מניין הגדול על פי אגורימא וכתוב תחתיו הי"ט כזה | ||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||
|
אמור כמה פעמים א' שפלה בתוך ה' של מעלה | ||||||||||||||||||||||||||
|
אם תיקח ה' פעמים ואז ממה תקח הט' ה' פעמים | ||||||||||||||||||||||||||
| ואם ד' פעמים ותאמר א' שהיא עשר במקום ה0' שלפניה לא תוכל ליקח ט' ד' פעמים מעשר' | |||||||||||||||||||||||||||
| ואם תקח א' ג' פעמים וישאר ב' במקום הב' שהם עשרים במקום ה0' הן לא תוכל ליקח הט' ג' פעמי' מעשרים | |||||||||||||||||||||||||||
| אכן קח הא' ב' פעמים וישאר במקום הה' ג' קח ב' מהג' והשב אחורנית מקום ה0' ויהיה עשרים וקח ט' ב' פעמים מעשרים וישאר ב' במקום ה0' וא' במקום הה' כתוב ב' שכל סך פעמ' מצאת י"ט בתוך מעלה אותה ב' כתוב תחת הט' ויהיה דמיונ' כך | |||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||
| והנה לא תוכל למצוא ט"א בב"א אכן השם ט"א אחרונית כזה | |||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||
| ואמור כמה פעמים א' בב"א וקח אותה כל כך מעט כדי שתוכל ליקח הט' גם כל כך ז' פעמים לא תוכל ליקח הט' מן הנשאר ז' פעמים כי מן הב"א ישאר ו"ח שעל הט' זהו ח"ה לא תשיג ליקח ממנו ז' פעמים ט' כי הוא עולה יותר אכן קח מן מ"ו פעמים מן הי"ב שעליה וישאר ו' במקו' הב' כזה | |||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||
| השב הט' אחורנית תחת הו' העליונה קח ו' פעמי' ט' מח"ו וישאר במקום הח' ד' ובמקום הו' א' כזה | |||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||
| ומונת עתה ו' פעמים ט"א במעלה שנייה כתוב ו' תחת הט' כזה | |||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||
| השב הט' אחורנית תחת הו' עליונה כזה | |||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||
| קח א' שפלה מד"א ז"פ וישאר במקום ז' במקום הד' ויהיה הדומיון כזה | |||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||
| קח הט' גם כן ז' פעמים מו"ז וישאר ג' במקום הו' וא' במקום הז' יכתוב הז' שמצאת' תחת הט' ויהיה הדומיון כך | |||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||
| וזה יורה לך שמצאת ט"א בתוך וח0"ה במעלה השלישית שהם מאות ו' במעלה השנייה שהם עשיריו' וז' במעלה ראשונה שהם אחדים וישארו ג"א שלא באו לחלק א"כ הן כמניין הגדול וז' פעמי' וג"א חלקים בשלימות ועל דרך זה תחלוק |
Proportions |
שער הערוך | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| הוא מועיל לידע מתוך חשבון ידוע חשבון נסתר | |||||||||||
|
כאשר ישאל השואל הנה ידוע לך שי"א תרנגולים נמכרים בכ"א פ' ועתה תשאל היאך ערך ח' תרנגולים | ||||||||||
| והנה אתן לך דרך אשר ממנו תדע הדרך כתוב י"א תרנגולים בטור ואחריו באותו טור כתוב הכ"א ובטור שנייה תחת הי"א כתוב כזה | |||||||||||
|
| ||||||||||
| ואמור כערך א"א על א"ב כן ערך א' על הנסתר | |||||||||||
| כפול ח' על הנסתר א"ב ויעלה חו"א | |||||||||||
| חלקיהא בא"א ותמצא כ"ו ט"ו פעמים א"א בתוך חו"א וישאר ג' שלא תוכל לחלוק | |||||||||||
| א"כ ח' תרנגולים ימכרו עבור ט"ו וג' שברים מן א"א כשלמין | |||||||||||
|
דומיון הנה קניתי י"ב אמות בגד בכ"ח דינ' וביקשת לידע כמה ערך של ז' אמות | ||||||||||
| כתוב י"ב והם האמות בטור ואחריו כתוב כ"ח והם דמים בטור שנייה כתוב הז' והם אמות אשר לא יתנם עד כ"ח וכותבם תחת הי"ב כזה | |||||||||||
|
| ||||||||||
| ויאמר כערך י"ב על ט"ו כן ערך הנסתר אל ז' | |||||||||||
| וככה תעשה כפל ח"ב בז' ויעלה וט"א | |||||||||||
| חלקם בב"א ויעלה ו"א וד' מי"ב בשלימים | |||||||||||
| א"כ ערך הז' אמות י"ז דינר וד' מי"ב בשלימי' | |||||||||||
|
דומיון הנה שכרתה שכיר ל' ימים במ"א דינר ולא עבד אתך אלא י"ז ימים ובקשת לידע כמה שכרו לאותו הימים | ||||||||||
| כתוב ל' והם הדמים אשר היה לו לעבוד בטור העליון ואחריו מ"א באותו הטור והם דמי השכיר אם עבד עמך כל הימים וכתוב בטור שנייה תחתיו הל' י"ז והם אשר עבד | |||||||||||
| Written according to agorima as follows | יכתוב אותם על פי אגורימא כזה | ||||||||||
|
| ||||||||||
| ואמר כערך ז"א אל א"ד כך ערך 0"ג אל הנסתר | |||||||||||
| כפול ז"א על א"ד והם זט"ו | |||||||||||
| חלקם ב0"ג ויעלה ג"ב וז' חלקים מ0"ג בשלמי' | |||||||||||
| א"כ אתה חייב לו מן העבודה אשר עבד אתך הי"ז ימים כ"ג דינר וז' פרוטות מל' בדינר | |||||||||||
| סליק שער הערוך | |||||||||||
Gelosia |
|||||||||||
| פי' זה הלוח | |||||||||||
| עשה עמו כפל בלי מחק כאשר רמזתי תקן | |||||||||||
|
|
ותאמר אם תרצה לידע כמה עולה ד'ה'ו' אז תכתוב כמו שכתבתי ז'ח'ט' | ||||||||||
|
|
ותאמר ז' פעמי' ד' הרי כ"ח | ||||||||||
|
|
ז' פעמי' ה' הרי ל"ה | ||||||||||
|
|
ז' פעמים ו' הרי מ"ב | ||||||||||
| וכן תעשה לכולם | |||||||||||
|
ח'ט'ד'ה'ד'ו' זה עולה | ||||||||||
| פי' זה הלוח לידע כמה הוה ב"פ ב' ג"פ ג' ד"פ ד' ה"פ ה' וכן כולם | |||||||||||
| סליק ספר צאיפור |
Appendix: Bibliography
Anonymous
Sefer Agris / Agrima / Sefer Ṣaifur / Ṣifur
Manuscripts:
- 1) Darmstadt, Hessisches Landes-und Hochschulbibliothek Cod. Or. 25/39 (IMHM: f 12695), ff. 71v-73r (14th century)
- Or. 25/39
- 2) Oxford, Bodleian Library MS Opp. 757/17 (IMHM: f 20981), ff. 322v-326v (cat. Neub. 2289,17); (16th century)
- 3) Warszaw, Żydowski Instytut Historyczny 288/2 (IMHM: f 12013), ff. 6r-22r (15th century)
The transcript is based mainly on manuscript Darmstadt
Bibliography:
- Steinschneider, Moritz. 1906. Mathematik bei den Juden, Band II: 1551-1840. Monatsschrift für die Geschichte und Wissenschaft des Judenthums 50, p. 213. repr.: ed. Gad Freudenthal, Hildesheim, Zürich, New York: Olms, 2014, p. 136.