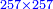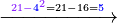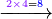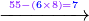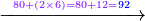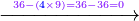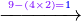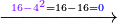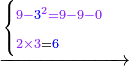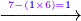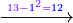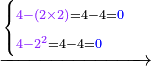Difference between revisions of "ספר ציפרא"
(→Chapter Three: Subtraction) |
(→Mantova, Comunità Ebraica MS ebr. 8/16) |
||
| Line 1: | Line 1: | ||
| + | {{#annotpage: time="1400-1500", peshat_title="00002046"}} | ||
| + | __TOC__ | ||
| + | <br> | ||
{| | {| | ||
|- | |- | ||
| | | | ||
| − | == Prologue == | + | == <span style=color:Green>Prologue</span> == |
| | | | ||
|- | |- | ||
| − | |[MS Paris, Séminaire Israélite de France (École Rabbinique) 158/1 (IMHM 4102) f. 197r, lines 1-16] | + | |<span style=color:Green>[MS Paris, Séminaire Israélite de France (École Rabbinique) 158/1 (IMHM 4102) f. 197r, lines 1-16]</span> |
| | | | ||
|- | |- | ||
| − | | | + | |This is a book on mathematics [called] Ṭeshifra. |
| − | |style="text-align:right;"|זה ספר חכמת התשבורת [הנקרא] טשיפרא סיבתו | + | |style="width:45%; text-align:right;"|זה ספר חכמת התשבורת [הנקרא] טשיפרא |
| + | |- | ||
| + | |Its cause: I myself, already from youth, my soul desired to acquire wisdom and to seek for arithmetic, if only I will find them in a book engraved by a pen of a skilled writer. For the reason that this is an external science, which is not found before us in Israel and the very least that is available is gathered inside the house in utmost discretion, my thoughts therefore aroused me to collect the sayings from any one that has them […] for recollection. | ||
| + | |style="text-align:right;"|סיבתו אני את לבי גם מנעורי גרסה נפשי לדעת חכמה ובקש חשבון ומי יתן ואמצאם בספר ויחקו ב''{{#annot:Ps45-2|494|kT7t}}עט סופר מהיר{{#annotend:kT7t}}'' ‫<ref group=note>תהילים מה, ב</ref> ולסבה שזאת היא חכמת החיצוניות לא נמצאת לפנינו בישראל והמיעוט שבמיעוט הנמצא נאסף אל תוך הבית בחדרי חדרים לכן העירוני רעיוני ללקט האמרים מיד כל אשר ימצא אתו ולכל[...] לזכרון | ||
|- | |- | ||
| − | | | + | |Before I start, I ask all its readers not to condemn me and say: what is it with you? Go study havayot d'Abbaye ve-Rabba! God forbid […]. Since I did not undertake it for any cause or purpose, but sharpening the mind and obtaining arithmetic by accident and learning and by the wittiness of Abbaye ve-Rabba primarily. On that said those who speak in parables: come to Ḥeshbon [Numbers 21, 27]. As is written in Maimonides, chapter five of the introduction to Tractate Avot: |
| − | |style="text-align:right;"|וקודם שאתחיל אבקש מיד כל רואיו לבל יכרעני לכף חובה ולומר מה לך פה כלך בהוייות דאביי ורבא חלילה וחס [לי'] לזרעא דאבא | + | |style="text-align:right;"|וקודם שאתחיל אבקש מיד כל רואיו לבל יכרעני לכף חובה ולומר מה לך פה כלך בהוייות דאביי ורבא חלילה וחס [לי'] לזרעא דאבא כי לא לקחתי זאת לשום עיקר ותכלית רק לחדד השכל ולבא בחשבון במקרה ושנייה ובחידוד דאביי ורבא בעצם וראשונה ועל זאת אמרו ''{{#annot:Num21-27|494|jpiM}}המושלים באו חשבון{{#annotend:jpiM}}'' ‫<ref group=note>במדבר כא, כז</ref> כמו שכתוב הרמבם ז"ל פרק חמישי מה[קדמת] ה פרקי אבות ‫<ref group=note>שמונה פרקים, פרק ה</ref> וז"ל |
|- | |- | ||
| − | | | + | |What has no benefit in it for that purpose – as the arithmetic problems, the Book of Conics, the numerous techniques for questions on geometry, the measuring of weights, and many like those – the intention in them is to sharpen the mind and train the rational faculty through methods of proof, until a person acquires the knowledge of demonstrative reasoning from other, and this will be his way whereby he will reach the knowledge of the truth of His existence, The Exalted. |
|style="text-align:right;"|ומה שאין בו תועלת לו בתכלית ההוא כשאלות החשבון וספר החר[וט]ים והתחבולות הרבות משאלות אל ההנד[ס]ה ומשיכת המשקלים והרבה כיוצא בא[ילו] יהיה הכוונה בהם לחדד השכל ולהרגיל כח השכלי בדרכי המופת עד שיגיע לו לאדם קניין ידיעת ההיקש המופתי מזולתו ויהיה לו זה הדרך שיגיע בה לידעת אמיתות מציאתו ית' עכל‫' | |style="text-align:right;"|ומה שאין בו תועלת לו בתכלית ההוא כשאלות החשבון וספר החר[וט]ים והתחבולות הרבות משאלות אל ההנד[ס]ה ומשיכת המשקלים והרבה כיוצא בא[ילו] יהיה הכוונה בהם לחדד השכל ולהרגיל כח השכלי בדרכי המופת עד שיגיע לו לאדם קניין ידיעת ההיקש המופתי מזולתו ויהיה לו זה הדרך שיגיע בה לידעת אמיתות מציאתו ית' עכל‫' | ||
| + | |- | ||
| + | |So, for my part it will not be inaccessible. | ||
| + | |style="text-align:right;"|גם מצדי לא יבצר | ||
|- | |- | ||
| | | | ||
| − | == Introduction == | + | == <span style=color:Green>Introduction</span> == |
| | | | ||
|- | |- | ||
| − | | | + | |With good luck |
| − | |style="text-align:right;"|בטוב גדא | + | |style="width:45%; text-align:right;"|‫<ref>109v</ref>בטוב גדא |
|- | |- | ||
| − | | | + | |I shall start this |
|style="text-align:right;"|אתחיל דא | |style="text-align:right;"|אתחיל דא | ||
|- | |- | ||
| − | | | + | |''Sefer Ṣifra'' |
| − | |style="text-align:right;"|ספר ציפרא | + | |style="text-align:right;"|ספר {{#annot:term|204,1813|YFdI}}ציפרא{{#annotend:YFdI}} |
|- | |- | ||
| − | | | + | |To know and explain the book of number that has 9 chapters. |
| − | |style="text-align:right;"|לידע | + | |style="text-align:right;"|לידע ולמפרש ספר המספר שיש בו ט' שערים |
|- | |- | ||
| − | | | + | |First I shall explain the teachings that the one who calculates should know, so that he can know all the calculations [practice]. |
| − | |style="text-align:right;"|ואפרש | + | |style="text-align:right;"|ואפרש תחלה הקבלות אשר צריך לידע {{#annot:term|230|Wgio}}המחשב{{#annotend:Wgio}} הוא {{#annot:term|230|7Y4T}}המפתח{{#annotend:7Y4T}} ויכול אח"כ לידע כל {{#annot:term|228,1200|SSQq}}החשבונו‫'{{#annotend:SSQq}} |
|- | |- | ||
| | | | ||
| − | === The Positional Decimal System === | + | === <span style=color:Green>The Positional Decimal System</span> === |
| | | | ||
|- | |- | ||
| + | !<span style=color:Green>The numerals</span> | ||
| | | | ||
| − | ==== The written | + | |- |
| − | + | |Know that you do not have any number [= numeral] smaller than one and you do not have any number [= numeral] greater than nine. | |
| + | |style="text-align:right;"|נידע כי אין לך {{#annot:term|204,1200|Zu7z}}חשבון{{#annotend:Zu7z}} {{#annot:term|341|ILMp}}קטן מן{{#annotend:ILMp}} א' שהוא <s>אח</s>אחד<br> | ||
| + | ואין לך חשבון שהוא {{#annot:term|342|k2sM}}גדול מן{{#annotend:k2sM}} ט‫' | ||
| + | |- | ||
| + | |By these nine numerals from א to ט [the nine first Hebrew letters] all numbers in the world [are formed] infinitely. How?: | ||
| + | |style="text-align:right;"|ובאילו ט' {{#annot:term|204,1332|b7Xx}}אותיו'{{#annotend:b7Xx}} מא' ועד ט' יש בו כל החשבונות שבעולם עד אין מספר | ||
| + | |- | ||
| + | !<span style=color:Green>The written ranks [= decimal places]</span> | ||
| | | | ||
|- | |- | ||
| | | | ||
| − | * | + | *<span style=color:Green>Units:</span> the first rank is called the rank of units, for as the digit is named so is its numerical value, meaning: 1 is one; 2 is two; and so on until 9, which is nine. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כיצד {{#annot:term|203,1316|wDMc}}מעלה{{#annotend:wDMc}} ראשונה היא נקראת {{#annot:term|287|vrY4}}מעלת היחידים{{#annotend:vrY4}} כי כאשר יקראו האות כך מניינם פי' א' אחד ב' שנים וכן עד ט' שהיא תשע |
|- | |- | ||
| | | | ||
| − | * | + | *<span style=color:Green>Tens:</span> |
| − | + | | | |
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | :<span style=color:Green>Zero – placeholder digit:</span> when you calculate ten you should write two ranks - 1 in the second rank |
| + | |style="text-align:right;"|וכשתחשוב עשרה אז צריך אתה לכתוב ב' מעלות א' במעלה שנייה | ||
|- | |- | ||
| | | | ||
| − | + | :{{#annot:definition|205,1813|FxB3}}and before it a numeral, which is a zero [lit. wheel], like this 0, that has no substance but is a place holder and a remnant of a thing ''like a wheel, [like stubble] before the wind'' [Psalms 83, 14]. | |
| − | == | + | |style="text-align:right;"|ולפניו ציפרא שהוא גלגל כזה 0 שאין בו ממש אלא שומר המעלות וזכר לדב' ''{{#annot:Ps83-14|494|JOTU}}כגלגל לפני רוח{{#annotend:JOTU}}'' ‫<ref group=note>תהלים פג, יד</ref>{{#annotend:FxB3}} |
| − | + | |- | |
| | | | ||
| + | :*א‎0 is 10. | ||
| + | |style="text-align:right;"|‫0א הוא י‫' | ||
|- | |- | ||
| | | | ||
| − | * | + | :*אא is 11. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אא הוא י"א |
|- | |- | ||
| | | | ||
| − | + | ::For the first א of the units is in the first rank and the second א is of the tens, hence 11. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כי הא' הראשונה מן היחידים שהיא במעלה ראשונה וא' שנייה היא מן העשיריו' הרי י"א |
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | :*בא is 12. |
| + | |style="text-align:right;"|בא הרי י"ב | ||
|- | |- | ||
| | | | ||
| − | :* | + | :*גא is 13. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|גא הרי י"ג |
|- | |- | ||
| | | | ||
| − | :* | + | :*and so on until טא which is 19. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וככה עד טא שהוא י"ט |
|- | |- | ||
| | | | ||
| − | * | + | :*ב‎0 is twenty. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|‫0ב הוא עשרים |
|- | |- | ||
| | | | ||
| − | :* | + | :*אב is twenty-one. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אב אחד ועשרים |
|- | |- | ||
| | | | ||
| − | :* | + | :*בב is twenty-two. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|בב שנים ועשרים |
|- | |- | ||
| | | | ||
| − | * | + | :*and so on until twenty-nine, which is טב. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וככה עד תשע ועשרי' שהוא טב |
|- | |- | ||
| | | | ||
| − | : | + | *<span style=color:Green>Hundreds:</span> the third rank is the rank of the hundreds, like this: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ושלישי הוא מעלות המאות כזה |
|- | |- | ||
| | | | ||
| − | * | + | :*א‎00 which is 100. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|‫00א שהוא ק‫' |
|- | |- | ||
| | | | ||
| − | * | + | :*ב‎00 which is 200. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|‫00ב הוא ר‫' |
|- | |- | ||
| | | | ||
| − | * | + | :*ג‎00 which is 300. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|‫00ג הוא ש‫' |
|- | |- | ||
| − | |and so on | + | | |
| − | |style="text-align:right;"|וככה עד | + | :*and so on until 9 hundred, which is ט‎00. |
| + | |style="text-align:right;"|וככה עד ט' מאות שהוא 00ט | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | :*אאא is 111. |
| + | |style="text-align:right;"|אאא הוא קי"א | ||
|- | |- | ||
| | | | ||
| − | : | + | :*א‎0א is 101. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|א0א הוא ק"א |
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | *<span style=color:Green>Thousands:</span> the fourth rank is the rank of thousands. |
| + | |style="text-align:right;"|ומעלה הרביעי' היא מעלת האלפי‫' | ||
|- | |- | ||
| − | | | + | | |
| − | + | :*א‎000 is one thousand. | |
| + | |style="text-align:right;"|‫000א הוא אלף | ||
|- | |- | ||
| | | | ||
| − | + | :*ב‎000 is two thousand. | |
| − | + | |style="text-align:right;"|‫000ב הוא ב' אלפי‫' | |
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | * | + | :*and so on until 9 thousand, which is ט‎000. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וככה עד ט' אלפים שהוא 000"ט |
|- | |- | ||
| | | | ||
| − | + | *<span style=color:Green>Tens of thousands:</span> the fifth rank is the rank of tens of thousands. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ומעלה החמישית היא {{#annot:term|346|I84k}}מעלת הרבבות{{#annotend:I84k}} שהוא י' אלפי‫' |
| − | {| | ||
|- | |- | ||
| − | |style= | + | | |
| − | + | *<span style=color:Green>Hundreds of thousands:</span> the sixth rank is the rank of hundreds of thousands. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וששה מעלה הוא מאה אלפי‫' |
| − | |||
|- | |- | ||
| | | | ||
| − | ::{| | + | *<span style=color:Green>Thousands of thousands:</span> the seventh rank is the rank of thousands of thousands [= millions]. |
| + | |style="text-align:right;"|ושביעי' {{#annot:term|350|U78S}}אלף אלפים{{#annotend:U78S}} | ||
|- | |- | ||
| − | | | + | |And so you can write endlessly. |
| + | |style="text-align:right;"|וככה עד אין מספר תוכל לכתוב | ||
|- | |- | ||
| − | | | + | |Every rank is ten times the preceding rank. |
| + | |style="text-align:right;"|וכל מעלה היא עשרה פעמי' יותר מן מעלה שלפניה | ||
|- | |- | ||
| − | | | + | | |
| − | + | :*Example: if one asks you: how much is 3020? | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואם ישאלך השואל 0ב0ג כמה הן |
| − | |||
|- | |- | ||
| − | |style="text-align:right;"| | + | | |
| + | ::Note that the zero has no numerical value but is a place holder, so the number is three thousand and twenty. | ||
| + | |style="text-align:right;"|תשיב אל לבך כי ה{{#annot:term|205,1472|tNw7}}גלגל{{#annotend:tNw7}} אין לו מניין רק {{#annot:term|233,2451|4yzF}}שומר המעלות{{#annotend:4yzF}} והנה החשבון עשרים וג' אלפים | ||
|- | |- | ||
| − | |style="text-align:right;"| | + | |Thus, I have explained to you the number and the meaning of the writing. |
| − | + | |style="text-align:right;"|והנה ביארתי לך מניין וענין הכתובי‫' | |
| − | |||
| − | |||
|- | |- | ||
| − | |style="text-align: | + | |1 2 3 4 5 6 7 8 9 0 10 20 30 |
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
|- | |- | ||
| − | | | + | |‫0ט||‫0א||0||ט||ח||ז||ו||ה||ד||ג||ב||א |
| + | |} | ||
|- | |- | ||
| − | |||
|} | |} | ||
| − | |||
| − | |||
|- | |- | ||
| − | |style="text-align:right;"| | + | | |
| + | |||
| + | == Chapter One: Multiplication of Units - Shortcuts == | ||
| + | |||
| + | |style="width: 45%; text-align:right;"|<big>שער הכפל הקטן</big> | ||
|- | |- | ||
| − | | | + | | colspan="2"| |
| + | *<math>\scriptstyle{\color{OliveGreen}{x,y<10\quad x\sdot y=\left[10\sdot\left[\left(x+y\right)-10\right]\right]+\left[\left[x-\left[\left(x+y\right)-10\right]\right]\sdot\left[y-\left[\left(x+y\right)-10\right]\right]\right]}}</math> | ||
|- | |- | ||
| − | |style="text-align:right;"| | + | |If you wish to know how much is the result of 9 times 8, or 5 times 9, or 6 times 9 and so on for all that is less than ten, I will teach you: |
| − | |} | + | |style="text-align:right;"|ואם תחפוץ לידע כמה עולה ט' {{#annot:term|243|W5M9}}פעמים{{#annotend:W5M9}} ח' או ה'פ'ז' או ו' פעמי' ט' [וכן כל כיוצא בזה שכל אחד פחות מעשרה]‫<ref>M om.</ref> אשכילך |
| − | |||
| − | |||
|- | |- | ||
| − | |style="text-align:right;"| | + | | |
| + | :*{{#annot:9×9|156|OZ9T}}If you wish to know how much is 9 times 9 | ||
| + | ::<math>\scriptstyle9\times9</math> | ||
| + | |style="text-align:right;"|אם תחפוץ לידע כמה ט’ פעמי' ט‫’{{#annotend:OZ9T}} | ||
|- | |- | ||
| − | |style="text-align:right;"| | + | | |
| + | ::Write like this: | ||
| + | |style="text-align:right;"|אז כתוב כזה | ||
|- | |- | ||
| − | |style="text-align: | + | | |
| − | + | ::{|class="wikitable" style="color:blue; text-align:center;" | |
| − | |||
|- | |- | ||
| | | | ||
| − | |||
| − | |||
{|style="margin-left: auto; margin-right: 0px;" | {|style="margin-left: auto; margin-right: 0px;" | ||
|- | |- | ||
| − | | | + | |9 |
|- | |- | ||
| − | | | + | |9 |
|} | |} | ||
|- | |- | ||
| + | |} | ||
| | | | ||
| − | :: | + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" |
|- | |- | ||
| − | | | + | | |
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
|- | |- | ||
| − | | | + | |ט |
|- | |- | ||
| − | | | + | |ט |
|} | |} | ||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |} |
|- | |- | ||
| − | | | + | | |
| − | + | ::Think by how much it exceeds over ten, it is 8. | |
| − | + | ::<math>\scriptstyle{\color{blue}{\left(9+9\right)-10=8}}</math> | |
| − | + | |style="text-align:right;"|ואח"כ חשוב כמה יותר על עשרה ח‫' | |
|- | |- | ||
| − | |style="text-align:right;"| | + | | |
| + | ::Write [it] next to the upper 9 to the left, like this: | ||
| + | |style="text-align:right;"|ואת' ח' כתוב אצל הט' העליונה ‫<ref>110r</ref>בצד שמאלית כזה | ||
|- | |- | ||
| − | |style="text-align: | + | | |
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
|- | |- | ||
| − | | | + | | |
| − | |||
| − | |||
{|style="margin-left: auto; margin-right: 0px;" | {|style="margin-left: auto; margin-right: 0px;" | ||
|- | |- | ||
| − | | | + | |8||9 |
|- | |- | ||
| − | | | + | | ||9 |
| + | |} | ||
|- | |- | ||
| − | |||
|} | |} | ||
| − | + | | | |
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
|- | |- | ||
| | | | ||
| − | |||
| − | |||
| − | |||
{|style="margin-left: auto; margin-right: 0px;" | {|style="margin-left: auto; margin-right: 0px;" | ||
|- | |- | ||
| − | | | + | |ח||ט |
| + | |- | ||
| + | | ||ט | ||
| + | |} | ||
|- | |- | ||
| − | |||
|} | |} | ||
|- | |- | ||
| | | | ||
| − | ::{| | + | ::Then, think by how much the upper 9 exceeds over the 8, you will find only 1. |
| + | ::<math>\scriptstyle{\color{blue}{9-8=1}}</math> | ||
| + | |style="text-align:right;"|ואח"כ חשוב כמה ט' העליונה {{#annot:term|420|3fXQ}}יותר על{{#annotend:3fXQ}} ח' ותמצא רק אחד | ||
|- | |- | ||
| − | | | + | | |
| + | ::Write this 1 next to the bottom 9: | ||
| + | |style="text-align:right;"|‫[אותו א’ כתוב בצד ט’ התחתונה‫]‫<ref>M om.</ref> | ||
|- | |- | ||
| − | | | + | | |
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
|- | |- | ||
| − | | | + | | |
| − | |||
| − | |||
| − | |||
{|style="margin-left: auto; margin-right: 0px;" | {|style="margin-left: auto; margin-right: 0px;" | ||
|- | |- | ||
| − | | | + | |8||9 |
| + | |- | ||
| + | | ||9 | ||
| + | |- | ||
| + | | ||1 | ||
| + | |} | ||
|- | |- | ||
| − | |||
|} | |} | ||
| − | + | | | |
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
{|style="margin-left: auto; margin-right: 0px;" | {|style="margin-left: auto; margin-right: 0px;" | ||
|- | |- | ||
| − | | | + | |ח||ט |
| + | |- | ||
| + | | ||ט | ||
| + | |- | ||
| + | | ||א | ||
| + | |} | ||
|- | |- | ||
| − | |||
|} | |} | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Then, think by how much the bottom 9 exceeds over the 8, it is 1. |
| + | ::<math>\scriptstyle{\color{blue}{9-8=1}}</math> | ||
| + | |style="text-align:right;"|וכמה ט' התחתונה יותר על הח' ותמצא רק אחד | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Write this 1 also next to the bottom 9, like this: |
| + | |style="text-align:right;"|‫[כתוב אותה א’ ג”כ בצד ט’ תחתונה כזה‫]‫<ref>M om.</ref> | ||
|- | |- | ||
| | | | ||
| − | + | ::{|class="wikitable" style="color:blue; text-align:center;" | |
| − | : | ||
| − | : | ||
| − | |style="text-align: | ||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | + | {|style="margin-left: auto; margin-right: 0px;" | |
| − | |||
| − | |||
|- | |- | ||
| − | |8|| | + | |8||9 |
|- | |- | ||
| − | | | + | | ||9 |
|- | |- | ||
| − | | | + | |1||1 |
| + | |} | ||
|- | |- | ||
|} | |} | ||
| Line 310: | Line 350: | ||
{|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
|- | |- | ||
| − | | | + | | |
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ח||ט | ||
|- | |- | ||
| − | | | + | | ||ט |
|- | |- | ||
| − | | | + | |א||א |
| + | |} | ||
|- | |- | ||
|} | |} | ||
| − | |||
|- | |- | ||
| | | | ||
| − | + | ::Think how much is 1 times 1, it is 1. | |
| − | + | ::<math>\scriptstyle{\color{blue}{1\times1=1}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ו{{#annot:term|185,1269|5Lxo}}תחשוב{{#annotend:5Lxo}} א'פ'א' היינו אחד |
|- | |- | ||
| | | | ||
| − | : | + | ::Write 1 before the 8, like this: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כתוב א' לפני הח' כזה |
|- | |- | ||
| | | | ||
| − | :: | + | ::{|class="wikitable" style="color:blue; text-align:center;" |
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | + | {|style="margin-left: auto; margin-right: 0px;" | |
|- | |- | ||
| − | | | + | |8||1 |
|- | |- | ||
| − | | | + | | ||9 |
|- | |- | ||
| − | | | + | |1||1 |
| − | | | + | |} |
| − | |||
|- | |- | ||
|} | |} | ||
| − | |||
| | | | ||
{|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
|- | |- | ||
| − | | | + | | |
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
|- | |- | ||
| − | |ח | + | |ח||א |
|- | |- | ||
| − | | | + | | ||ט |
|- | |- | ||
| + | |א||א | ||
|} | |} | ||
| − | |||
|- | |- | ||
| − | | | + | |} |
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Erase the bottom and the upper 9, so they are eighty-one. |
| + | |style="text-align:right;"|‫[ומחוק הט' למטה ולמעלה]‫<ref>M om.</ref> ויהיה אחד ושמונים | ||
|- | |- | ||
| − | | | + | | colspan="2"| |
| − | : | + | ::<span style=color:Green>[Illustration of the procedure:]</span> |
| − | + | ::{| | |
| − | {| | ||
|- | |- | ||
| − | |style=" | + | |<span style="color:purple">9</span>||rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{Purple}{\left(9+9\right)-10}}={\color{blue}{8}}}</math>||<span style="color:#0000FF>8</span><span style="color:purple">9</span>||rowspan="3"|<math>\scriptstyle\xrightarrow{\begin{cases}\scriptstyle{\color{Purple}{9-8}}={\color{blue}{1}}\\\scriptstyle{\color{Purple}{9-8}}={\color{blue}{1}}\end{cases}}</math>||89||rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{Purple}{1\sdot1}}={\color{blue}{1}}}</math>||8<span style="color:#0000FF>1</span> |
|- | |- | ||
| − | |style=" | + | |<span style="color:purple">9</span>|| <span style="color:purple">9</span>|| 9|| 9 |
| − | | | ||
|- | |- | ||
| + | | || ||<span style="color:#0000FF>11</span>||11 | ||
|} | |} | ||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | | |
| + | :*{{#annot:9×8|156|FWoZ}}Also if you want to know how much is 9 times 8 | ||
| + | ::<math>\scriptstyle9\times8</math> | ||
| + | |style="text-align:right;"|וכן אם תרצה לידע כמה ט' פעמים ח‫'{{#annotend:FWoZ}} | ||
|- | |- | ||
| − | | | + | | |
| + | ::Write like this: | ||
| + | |style="text-align:right;"|כתוב כזה | ||
|- | |- | ||
| − | | | | + | | |
| − | + | ::{|class="wikitable" style="color:blue; text-align:center;" | |
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | |||
{|style="margin-left: auto; margin-right: 0px;" | {|style="margin-left: auto; margin-right: 0px;" | ||
|- | |- | ||
| − | | | + | |9 |
| + | |- | ||
| + | |8 | ||
| + | |} | ||
|- | |- | ||
| − | |||
|} | |} | ||
| − | + | | | |
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
{|style="margin-left: auto; margin-right: 0px;" | {|style="margin-left: auto; margin-right: 0px;" | ||
|- | |- | ||
| − | | | + | |ט |
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |ח |
|} | |} | ||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| − | |||
|} | |} | ||
| − | |||
| − | |||
|- | |- | ||
| − | |style="text-align:right;"| | + | | |
| + | ::Think by how much 9+8 exceeds over ten, it is 7. | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(9+8\right)-10=7}}</math> | ||
| + | |style="text-align:right;"|וחשוב כמה ח'ט' יותר על עשרה דהיינו ז‫' | ||
|- | |- | ||
| − | |style="text-align:right;"| | + | | |
| − | + | ::Write the 7 next to the 9, like this: | |
| + | |style="text-align:right;"|כתוב הז' אצל הט' כזה | ||
|- | |- | ||
| | | | ||
| − | + | ::{|class="wikitable" style="color:blue; text-align:center;" | |
| − | |||
|- | |- | ||
| | | | ||
| − | |||
| − | |||
{|style="margin-left: auto; margin-right: 0px;" | {|style="margin-left: auto; margin-right: 0px;" | ||
|- | |- | ||
| − | | | + | |7||9 |
|- | |- | ||
| − | | | + | | ||8 |
|} | |} | ||
| − | + | |- | |
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
|- | |- | ||
| | | | ||
| − | :: | + | {|style="margin-left: auto; margin-right: 0px;" |
|- | |- | ||
| − | | | + | |ז||ט |
|- | |- | ||
| − | + | | ||ח | |
| + | |} | ||
|- | |- | ||
| − | |||
|} | |} | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | + | ::Think by how much the 9 exceeds over the 7, it is 2. | |
| − | + | ::<math>\scriptstyle{\color{blue}{9-7=2}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וחשוב כמה ט' {{#annot:term|996,1352|VP6t}}עודף על{{#annotend:VP6t}} ז' דהיינו ב‫' |
|- | |- | ||
| | | | ||
| − | : | + | ::By how much the 8 exceeds over the 7, [it is] 1. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{8-7=1}}</math> |
| + | |style="text-align:right;"|וכמה הח' עודף על ז' א‫' | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::Write like this: |
| + | |style="text-align:right;"|‫[וכתוב כזה]‫<ref>M om.</ref> | ||
|- | |- | ||
| − | | | + | | |
| − | + | ::{|class="wikitable" style="color:blue; text-align:center;" | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | + | {|style="margin-left: auto; margin-right: 0px;" | |
| − | : | ||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |7||9 |
| − | |||
| − | | | ||
|- | |- | ||
| − | | | + | | ||8 |
| − | |||
|- | |- | ||
| − | | | + | |1||2 |
|} | |} | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| + | |} | ||
| | | | ||
| − | + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | |
| − | |style="text-align: | ||
|- | |- | ||
| | | | ||
| − | :: | + | {|style="margin-left: auto; margin-right: 0px;" |
|- | |- | ||
| − | | | + | |ז||ט |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | | ||ח |
| − | |||
| − | |||
| − | | | ||
|- | |- | ||
| − | | | + | |א||ב |
| − | + | |} | |
|- | |- | ||
| − | |||
|} | |} | ||
| − | |||
|- | |- | ||
| − | |||
| | | | ||
| + | ::Multiply 1 by 2, it is 2. | ||
| + | ::<math>\scriptstyle{\color{blue}{1\times2=2}}</math> | ||
| + | |style="text-align:right;"|תכפול א' על ב' היינו ב‫' | ||
|- | |- | ||
| | | | ||
| − | + | ::Write this 2 before the 7, like this: | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אות' ב' כתוב קודם הז' כזה |
|- | |- | ||
| | | | ||
| − | + | ::{|class="wikitable" style="color:blue; text-align:center;" | |
| − | : | ||
| − | |style="text-align: | ||
|- | |- | ||
| | | | ||
| − | |style=" | + | {|style="margin-left: auto; margin-right: 0px;" |
| + | |- | ||
| + | |7||2 | ||
| + | |- | ||
| + | | ||8 | ||
|- | |- | ||
| + | |1||2 | ||
|} | |} | ||
| − | |||
|- | |- | ||
| + | |} | ||
| | | | ||
| − | + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | |
| − | = | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | |||
| − | |||
{|style="margin-left: auto; margin-right: 0px;" | {|style="margin-left: auto; margin-right: 0px;" | ||
|- | |- | ||
| − | | | + | |ז||ב |
| + | |- | ||
| + | | ||ח | ||
| + | |- | ||
| + | |א||ב | ||
| + | |} | ||
|- | |- | ||
| − | |||
|} | |} | ||
| − | |||
|- | |- | ||
| | | | ||
| + | ::We find that 9 times 8 is 72. | ||
| + | |style="text-align:right;"|נמצא ט' פעמי' ח' יהיה ע"ב | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | ::<span style=color:green>[Illustration of the procedure:]</span> | ||
::{| | ::{| | ||
|- | |- | ||
| − | | | + | |<span style="color:purple">9</span>||rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{Purple}{\left(9+8\right)-10}}={\color{blue}{7}}}</math>||<span style="color:#0000FF>7</span><span style="color:purple">9</span>||rowspan="3"|<math>\scriptstyle\xrightarrow{\begin{cases}\scriptstyle{\color{Purple}{9-7}}={\color{blue}{2}}\\\scriptstyle{\color{Purple}{8-7}}={\color{blue}{1}}\end{cases}}</math>||79||rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{Purple}{1\sdot2}}={\color{blue}{2}}}</math>||7<span style="color:#0000FF>2</span> |
|- | |- | ||
| − | | | + | |<span style="color:purple">8</span>|| <span style="color:purple">8</span>|| 8|| 8 |
|- | |- | ||
| − | | || | + | | || ||<span style="color:#0000FF>12</span>||12 |
|} | |} | ||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| − | |style="text-align:right;"| | + | | |
| + | :*{{#annot:6×6|156|sgDh}}Example: if you wish to know how much is 6 times 6 | ||
| + | ::<math>\scriptstyle6\times6</math> | ||
| + | |style="text-align:right;"|‫<ref>MS Paris 1088, 4v: supplement</ref>דומיון אם תחפוץ לידע כמה ו' פעם ו‫'{{#annotend:sgDh}} | ||
|- | |- | ||
| − | |style="text-align:right;"| | + | | |
| + | ::Write the 6 beneath the 6, like this: | ||
| + | |style="text-align:right;"|אז כתו' הו' תחת הו' כזה | ||
|- | |- | ||
| − | |style="text-align: | + | | |
| − | + | ::{|class="wikitable" style="color:blue; text-align:center;" | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | | |
| − | |style=" | + | {|style="margin-left: auto; margin-right: 0px;" |
|- | |- | ||
| − | | | + | |6 |
| − | |||
|- | |- | ||
| − | | | + | |6 |
| − | | | + | |} |
|- | |- | ||
| + | |} | ||
| | | | ||
| − | + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | |
| − | |style="text-align: | ||
|- | |- | ||
| | | | ||
| − | |||
{|style="margin-left: auto; margin-right: 0px;" | {|style="margin-left: auto; margin-right: 0px;" | ||
|- | |- | ||
| − | | | + | |ו |
|- | |- | ||
| − | | | + | |ו |
|} | |} | ||
|- | |- | ||
|} | |} | ||
| − | |||
|- | |- | ||
| − | | | + | | |
| + | ::Say: by how much the two 6 exceed over 10, it is 2. | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(6+6\right)-10=2}}</math> | ||
| + | |style="text-align:right;"|ואז תאמר כמה השני ו' {{#annot:term|420|Qkw0}}למעלה מ{{#annotend:Qkw0}}י' זהו ב‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Write this 2 after the 6, like this: | ||
| + | |style="text-align:right;"|ואותו ב' תכתו' לאחר הו' כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |2||6 | ||
|- | |- | ||
| − | | | + | | ||6 |
| + | |} | ||
|- | |- | ||
| − | |||
|} | |} | ||
| − | {| | + | | |
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
|- | |- | ||
| | | | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
{|style="margin-left: auto; margin-right: 0px;" | {|style="margin-left: auto; margin-right: 0px;" | ||
|- | |- | ||
| − | | | + | |ב||ו |
|- | |- | ||
| − | + | | ||ו | |
| + | |} | ||
|- | |- | ||
| − | |||
|} | |} | ||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Then, say: by how much the 6 exceeds over 2, it is 4. |
| − | + | ::<math>\scriptstyle{\color{blue}{6-2=4}}</math> | |
| − | + | |style="text-align:right;"|ואז תאמ' כמה הו' {{#annot:term|420,1399|do7f}}יתיר על{{#annotend:do7f}} הב' זהו ד‫' | |
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | ::The second 6 also exceeds over 2 by 4. |
| + | ::<math>\scriptstyle{\color{blue}{6-2=4}}</math> | ||
| + | |style="text-align:right;"|והו' השנייה נמי למעל' מב' זהו ד‫' | ||
|- | |- | ||
| | | | ||
| − | {| | + | ::Multiply 4 times 4, it is 16, meaning sixteen. |
| + | ::<math>\scriptstyle{\color{blue}{4\times4=16}}</math> | ||
| + | |style="text-align:right;"|ואז {{#annot:term|185,1230|TNoO}}תכפול{{#annotend:TNoO}} ד' פעמי' ד' זהו ו"א פי' י"ו | ||
|- | |- | ||
| − | |<math>\scriptstyle{\color{blue}{ | + | | |
| + | ::Add the 1 to the 2, because the 2 is in the second rank, it is 3. | ||
| + | ::<math>\scriptstyle{\color{blue}{2+1=3}}</math> | ||
| + | |style="text-align:right;"|ואז תכתוב שים הא' על הב' כי הב' מעלה שניי' ויהיה ג‫' | ||
|- | |- | ||
| | | | ||
| − | + | ::There is also 6, write the 6 before the 3, like this: | |
| + | |style="text-align:right;"|ועוד הוה ו' ואות' ו' תכתו' לפני הג' כזה | ||
|- | |- | ||
| − | |style="text-align: | + | | |
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
|- | |- | ||
| − | |||
| − | |||
| | | | ||
| − | {|style=" | + | {|style="margin-left: auto; margin-right: 0px;" |
|- | |- | ||
| − | | | + | |3||6 |
|- | |- | ||
| − | + | | ||6 | |
|} | |} | ||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| − | |||
|} | |} | ||
| − | + | | | |
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
|- | |- | ||
| | | | ||
| − | {|style=" | + | {|style="margin-left: auto; margin-right: 0px;" |
|- | |- | ||
| − | | | + | |ג||ו |
| − | |||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | | ||ו |
|} | |} | ||
| − | |||
| − | |||
|- | |- | ||
| − | |||
|} | |} | ||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | + | ::We find that 6 times 6 is 36. | |
| + | |style="text-align:right;"|נמצא שו' פעמ' ו' עולה ו"ג פי' ל"ו | ||
|- | |- | ||
| − | | | + | | colspan="2"| |
| + | ::<span style=color:green>[Illustration of the procedure:]</span> | ||
| + | ::{| | ||
|- | |- | ||
| − | |style=" | + | |<span style="color:purple">6</span>||rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{Purple}{\left(6+6\right)-10}}={\color{blue}{2}}}</math>||<span style="color:#0000FF>2</span><span style="color:purple">6</span>||rowspan="3"|<math>\scriptstyle\xrightarrow{\begin{cases}\scriptstyle{\color{Purple}{6-2}}={\color{blue}{4}}\\\scriptstyle{\color{Purple}{6-2}}={\color{blue}{4}}\end{cases}}</math>||[26]||rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{Purple}{20+\left(4\sdot4\right)}}={\color{blue}{36}}}</math>||<span style="color:#0000FF>36</span> |
| − | |} | ||
| − | | | ||
| − | |||
|- | |- | ||
| − | |style=" | + | |<span style="color:purple">6</span>|| <span style="color:purple">6</span>|| [6]|| 6 |
|- | |- | ||
| − | |style=" | + | | || ||[<span style="color:#0000FF>44</span>]|| |
|} | |} | ||
| − | |||
| − | |||
|- | |- | ||
| − | |style="text-align:right;"| | + | |Always do as this. |
| + | |style="text-align:right;"|וכן עשה לעולם‫<ref>MS Paris 1088, 4v: end of the supplement</ref> | ||
|- | |- | ||
| − | |style="text-align:right;"| | + | |Multiply always the excess of this by the excess of this and write the result before the excess [of the sum of the multipliers over ten], then you will become wise in all that you do. |
| − | + | |style="text-align:right;"|וכן לעולם תכפול המותר מזה ומזה והעולה כתו' קודם העודף עליו ואז תשכיל בכל אשר תעשה | |
| − | |||
|- | |- | ||
| − | | | + | | colspan="2"| |
| − | { | + | *<math>\scriptstyle{\color{OliveGreen}{x,y<10\quad x\sdot y=\left[10\sdot\left[\left(x+y\right)-10\right]\right]+\left[\left(10-y\right)\sdot\left(10-x\right)\right]}}</math> |
|- | |- | ||
| − | |style="text-align:right;"| | + | |Another example that I translated from a '''Latin book''' |
| − | + | |style="text-align:right;"|‫<ref>MS Paris 158, 198r: supplement</ref>דמיון אחר שהעתקתי מספר גלחות | |
| − | |||
| − | |||
|- | |- | ||
| − | |style="text-align:right;"| | + | |Sum together the two numerals, subtract a ten, then write the small number. |
| − | + | |style="text-align:right;"|חברם יחד הב' אותיות והשליך עשרה והמניין הקטון כתוב | |
| − | |||
| − | |||
|- | |- | ||
| − | |style="text-align:right;"| | + | |Multiply together the differences of each from ten and write [the product] before the small number that you have. |
| − | + | |style="text-align:right;"|ואח"כ תכפול יחד החשבון שיש מכל אחד עד עשרה וכתוב זה לפני המנין הקטן שבידך | |
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |If this product is of two ranks, add the second rank to the number that you have. |
| − | + | |style="text-align:right;"|ואם בא לידך מהכפל הזה ב' מעלות אז תחבר המעלה שנייה אל המניין שבידך | |
| − | |||
| − | |style="text-align:right;"| | ||
|- | |- | ||
| − | | | + | |As these calculations that are written in front of you, from which you will become wiser: |
| − | | | + | |style="text-align:right;"|כגון אילו החשבונות החרו[ת]ים לפניך ממנו תשכיל ותחכם עוד |
|- | |- | ||
| | | | ||
| − | : | + | ::{|class="wikitable" style="color: blue; text-align:center;" |
| − | |style="text-align: | ||
| − | |||
|- | |- | ||
| − | | | + | |colspan="2"|<math>\scriptstyle{\color{blue}{8\times9}}</math>||colspan="2"|<math>\scriptstyle{\color{blue}{7\times8}}</math>||colspan="2"|<math>\scriptstyle{\color{blue}{6\times7}}</math> |
| − | |||
| − | | | ||
|- | |- | ||
| − | | | + | |8||2||7||3||6||4 |
| − | |||
|- | |- | ||
| − | | | + | |9||1||8||2||7||3 |
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |colspan="2"|72||colspan="2"|56||colspan="2"|42 |
| − | | | ||
| − | |||
|- | |- | ||
| + | |} | ||
| | | | ||
| − | + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | |
| − | | | ||
| − | |||
|- | |- | ||
| − | | | + | |8||2||7||3||6||4 |
|- | |- | ||
| − | | | + | |9||1||8||2||7||3 |
| − | | | ||
|- | |- | ||
| − | | | + | |colspan="2"|72||colspan="2"|56||colspan="2"|42 |
| − | |||
| − | |||
| − | |||
|- | |- | ||
| − | |||
|} | |} | ||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | | colspan="2"| |
| + | *<math>\scriptstyle{\color{OliveGreen}{x<y<10\quad x\sdot y=\left(x\sdot10\right)-\left[x\sdot\left(10-y\right)\right]}}</math> | ||
|- | |- | ||
| − | |style="text-align:right;"| | + | |Or in this way: write zero 0 before the smaller of the two numerals. |
| − | + | |style="text-align:right;"|או כלך לדרך זו כתוב לפני המניין קטון שבשני אותיות גלגל כזה 0 | |
| − | |||
| − | |||
|- | |- | ||
| − | |style="text-align:right;"| | + | | |
| + | :*{{#annot:7×8|156|B4fP}}Example: if you wish to know [how much is] 7 times 8. | ||
| + | ::<math>\scriptstyle7\times8</math> | ||
| + | |style="text-align:right;"|כגון את"ל ז'פ'ח‫'{{#annotend:B4fP}} | ||
|- | |- | ||
| − | |style="text-align:right;"| | + | | |
| − | + | ::Write like this: 70 | |
| − | + | |style="text-align:right;"|כתוב כזה 0ז | |
| − | |||
|- | |- | ||
| | | | ||
| − | + | ::Subtract from it the product of the difference of the greater numeral from ten by the smaller numeral. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וקח ממנו מה שבא מן הכפל שתכפול המניין מאות הגדול עד עשרה עם האות הקטן |
|- | |- | ||
| | | | ||
| − | {| | + | ::As what is infront of you: say 7 times 2 are 14. |
| + | ::Subtract 14 from 70, the remainder is 56. | ||
| + | ::<math>\scriptstyle{\color{blue}{7\times8=\left(7\sdot10\right)-\left[7\sdot\left(10-8\right)\right]=70-\left(7\sdot2\right)=70-14=56}}</math> | ||
| + | |style="text-align:right;"|כגון מה שלפניך אמור ז'פ'ב' הם ד"א<br> | ||
| + | ותקח ד"א מן 0"ז ונשאר ו"ה | ||
|- | |- | ||
| − | | | + | | |
| + | :*Such as these: | ||
| + | |style="text-align:right;"|וכגון אילו‫<ref>MS Paris 158, 198r: end of the supplement</ref> | ||
|- | |- | ||
| − | | | + | | |
| − | + | ::{|class="wikitable" style="color: blue; text-align:center;" | |
| − | + | |- | |
| − | + | |colspan="2"|<math>\scriptstyle{\color{blue}{8\times8}}</math>||colspan="2"|<math>\scriptstyle{\color{blue}{6\times7}}</math>||colspan="2"|<math>\scriptstyle{\color{blue}{4\times9}}</math>||colspan="2"|<math>\scriptstyle{\color{blue}{5\times8}}</math> | |
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |8||0||6||0||4||0||5||0 |
| − | | | ||
|- | |- | ||
| − | | | + | |8||2||7||3||9||1||8||2 |
| − | | | ||
|- | |- | ||
| − | | | + | |colspan="2"|64||colspan="2"|42||colspan="2"|36||colspan="2"|40 |
| − | | | ||
|- | |- | ||
|} | |} | ||
| − | |||
| − | |||
| | | | ||
| − | + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | |
| − | = | ||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |ח||‫0||‫ו||0||ד||‫0||‫ה||0 |
| − | | | ||
|- | |- | ||
| − | | | + | |ח||ב||ז||ג||ט||א||ח||ב |
| − | | | ||
|- | |- | ||
| − | | | + | |colspan="2"|דו||colspan="2"|בד||colspan="2"|וג||colspan="2"|‫0ד |
| − | | | ||
|- | |- | ||
| − | | | + | |} |
| − | |||
|- | |- | ||
| − | | | + | | colspan="2"| |
| − | + | *<math>\scriptstyle{\color{OliveGreen}{x+y<10\quad x\sdot y=10+\left[\left(x-1\right)\sdot\left(y-1\right)\right]-\left[11\left(x+y\right)\right]}}</math> | |
|- | |- | ||
| − | | | + | |If you want to know and calculate how much is 3 times 3, or 4 times 4, et cetera, which even their sum does not reach ten, I will teach you how to do so: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואם תרצה לחשוב כמה ג' פעמי' ג' ד'פ'ד' וכן כל כיוצא בזה שאפילו {{#annot:term|388,1678|fjvy}}צירופו{{#annotend:fjvy}} אינו מגיע לעשרה אשכילך לעשות |
|- | |- | ||
| − | | | + | |Lend to it as much as needed so that it will be one more than ten. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|שתלוה לו <s>פ</s> כמה שצריך שיהיה בכל פעם א' יותר מי‫' |
|- | |- | ||
| − | | | + | |When your calculation is complete, subtract what you have lent to it and the required remains. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וככלות חשבונך קח [מה שהלוית לו]‫<ref>M: מהם</ref> וישאר מתכונ‫' |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*{{#annot:4×4|156|ynhp}}Example: 4 times 4. |
| + | ::<math>\scriptstyle4\times4</math> | ||
| + | |style="text-align:right;"|דמיון ד'פ'ד‫'{{#annotend:ynhp}} | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Like this: |
| + | |style="text-align:right;"|כזה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align: | + | ::{|class="wikitable" style="color:blue; text-align:center;" |
|- | |- | ||
| | | | ||
| − | |style=" | + | {|style="margin-left: auto; margin-right: 0px;" |
|- | |- | ||
| − | | | + | |4 |
| − | |||
|- | |- | ||
| − | | | + | |4 |
| − | | | + | |} |
|- | |- | ||
| + | |} | ||
| | | | ||
| − | |style="text-align: | + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" |
|- | |- | ||
| | | | ||
| − | |style=" | + | {|style="margin-left: auto; margin-right: 0px;" |
|- | |- | ||
| − | | | + | |ד |
| − | |||
|- | |- | ||
| − | | | + | |ד |
| − | | | + | |} |
|- | |- | ||
| − | | | + | |} |
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Lend to it 3, so that it becomes 11. |
| + | ::<math>\scriptstyle{\color{blue}{4+4+\left[11-\left(4+4\right)\right]=4+4+3=11}}</math> | ||
| + | |style="text-align:right;"|והנה תלוה לו ג' שיעלה י"א | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Subtract ten, 1 remains in your hand. |
| + | ::<math>\scriptstyle{\color{blue}{11-10=1}}</math> | ||
| + | |style="text-align:right;"|ו{{#annot:term|181,1265|839i}}השלך{{#annotend:839i}} עשרה וישאר בידך א‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Write it like this: |
| + | |style="text-align:right;"|ורשום כזה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align: | + | ::{|class="wikitable" style="color:blue; text-align:center;" |
|- | |- | ||
| | | | ||
| − | |style=" | + | {|style="margin-left: auto; margin-right: 0px;" |
|- | |- | ||
| − | | | + | |1||4 |
| − | | | ||
|- | |- | ||
| − | | | + | | ||4 |
| − | | | + | |} |
|- | |- | ||
| + | |} | ||
| | | | ||
| − | |style="text-align: | + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" |
|- | |- | ||
| | | | ||
| − | |style=" | + | {|style="margin-left: auto; margin-right: 0px;" |
|- | |- | ||
| − | | | + | |א||ד |
| − | | | ||
|- | |- | ||
| − | | | + | | ||ד |
| − | | | + | |} |
|- | |- | ||
| − | | | + | |} |
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Think by how much the first 4 exceeds over the 1, that is to say 3. |
| + | ::<math>\scriptstyle{\color{blue}{4-1=3}}</math> | ||
| + | |style="text-align:right;"|ואח"כ חשוב כמה ד' הראשו' יותר על הא' הוי אומר ג‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::So, the second 4 also exceeds by 3. |
| + | ::<math>\scriptstyle{\color{blue}{4-1=3}}</math> | ||
| + | |style="text-align:right;"|וכן ד' השנייה ג"כ יותר ג‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align: | + | ::{|class="wikitable" style="color:blue; text-align:center;" |
|- | |- | ||
| | | | ||
| − | |style=" | + | {|style="margin-left: auto; margin-right: 0px;" |
| − | |||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |1||4 |
| − | | | ||
|- | |- | ||
| − | | | + | | ||4 |
| − | | | ||
|- | |- | ||
| − | | | + | |3||3 |
| − | | | + | |} |
|- | |- | ||
| + | |} | ||
| | | | ||
| − | |style="text-align: | + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" |
|- | |- | ||
| | | | ||
| − | |style=" | + | {|style="margin-left: auto; margin-right: 0px;" |
|- | |- | ||
| − | | | + | |א||ד |
| − | | | ||
|- | |- | ||
| − | | | + | | ||ד |
| − | | | ||
|- | |- | ||
| − | | | + | |ג||ג |
| − | | | + | |} |
|- | |- | ||
|} | |} | ||
| − | |||
|- | |- | ||
| | | | ||
| − | + | ::Multiply 3 by 3, it is 9. | |
| − | + | ::<math>\scriptstyle{\color{blue}{3\times3=9}}</math> | |
| − | + | |style="text-align:right;"|כפול ג' על ג' הרי ט‫' | |
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Write the 9 before the 1, like this: |
| + | |style="text-align:right;"|כתוב הט' לפני הא' כזה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align: | + | ::{|class="wikitable" style="color:blue; text-align:center;" |
|- | |- | ||
| | | | ||
| − | |style=" | + | {|style="margin-left: auto; margin-right: 0px;" |
|- | |- | ||
| − | | | + | |1||9 |
| − | | | ||
|- | |- | ||
| − | | | + | | ||4 |
| − | | | + | |} |
|- | |- | ||
| + | |} | ||
| | | | ||
| − | |style="text-align: | + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" |
|- | |- | ||
| | | | ||
| − | |style=" | + | {|style="margin-left: auto; margin-right: 0px;" |
|- | |- | ||
| − | | | + | |א||ט |
| − | | | ||
|- | |- | ||
| − | | | + | | ||ד |
| − | | | + | |} |
|- | |- | ||
| − | | | + | |} |
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Subtract 3 that you have lent to it and 16 remain, which are sixteen. |
| + | ::<math>\scriptstyle{\color{blue}{19-3=16}}</math> | ||
| + | |style="text-align:right;"|והנה קח ג' שהלוית וישאר ו'א' [והם י"ו‫]‫<ref>M om.</ref> | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::You will find that your calculation is correct. |
| + | |style="text-align:right;"|ותמצא חשבונך {{#annot:term|2079,2250|HkVh}}מכוון{{#annotend:HkVh}} | ||
|- | |- | ||
| | | | ||
| − | |style="text-align: | + | ::{|class="wikitable" style="color:blue; text-align:center;" |
|- | |- | ||
| | | | ||
| − | |style=" | + | {|style="margin-left: auto; margin-right: 0px;" |
|- | |- | ||
| − | | | + | |1||6 |
| − | | | ||
|- | |- | ||
| − | | | + | | ||4 |
| − | | | + | |} |
|- | |- | ||
| + | |} | ||
| | | | ||
| − | |style="text-align: | + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | {|style="margin-left: auto; margin-right: 0px;" |
| + | |- | ||
| + | |א||ו | ||
| + | |- | ||
| + | | ||ד | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | ::<span style=color:green>[Illustration of the procedure:]</span> | ||
| + | ::{| | ||
| + | |- | ||
| + | |<span style="color:purple">4</span>||rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{Purple}{\left[4+4+\left[11-\left(4+4\right)\right]\right]-10}}={\color{blue}{1}}}</math>||<span style="color:#0000FF>1</span><span style="color:purple">4</span>||rowspan="3"|<math>\scriptstyle\xrightarrow{\begin{cases}\scriptstyle{\color{Purple}{4-1}}={\color{blue}{3}}\\\scriptstyle{\color{Purple}{4-1}}={\color{blue}{3}}\end{cases}}</math>||14||rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{Purple}{3\sdot3}}={\color{blue}{9}}}</math>||1<span style="color:#0000FF>9</span>||rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{Purple}{9-\left[11-\left(4+4\right)\right]}}={\color{blue}{6}}}</math>||1<span style="color:#0000FF>6</span> | ||
| + | |- | ||
| + | |<span style="color:purple">4</span>|| <span style="color:purple">4</span>|| 4|| 4|| 4 | ||
| + | |- | ||
| + | | || ||<span style="color:#0000FF>33</span>|| || || | ||
| + | |} | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | *<math>\scriptstyle{\color{OliveGreen}{x+y<10\quad x\sdot y=\left[\left(10-y\right)\sdot\left(10-x\right)\right]-\left[10\sdot\left[10-\left(x+y\right)\right]\right]}}</math> | ||
| + | |- | ||
| + | |Another property: if you want to know how much is 3 times 3, or 4 times 4, et cetera, which even their sum does not reach ten, I will teach: | ||
| + | |style="text-align:right;"|עניין אחר אם תרצה לידע כמה ג"פ"ג או ד"פ"ד וכן כל כיוצא בזה שאפי' צירופו אינו מגיע לעשרה אשכילך | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*{{#annot:4×4|156|yXdJ}}I will teach you an example: if you want to multiply 4 times 4. |
| + | ::<math>\scriptstyle4\times4</math> | ||
| + | |style="text-align:right;"|דומיון אם תרצה לחשוב ד'פ'ד‫’{{#annotend:yXdJ}} | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Write it like this: |
| + | |style="text-align:right;"|אז תכתוב אותו כזה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align: | + | ::{|class="wikitable" style="color:blue; text-align:center;" |
|- | |- | ||
| | | | ||
| − | |style=" | + | {|style="margin-left: auto; margin-right: 0px;" |
|- | |- | ||
| + | |4 | ||
| + | |- | ||
| + | |4 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| | | | ||
| − | |style="text-align: | + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" |
|- | |- | ||
| | | | ||
| − | |style=" | + | {|style="margin-left: auto; margin-right: 0px;" |
|- | |- | ||
| − | | | + | |ד |
| − | |||
|- | |- | ||
| − | | | + | |ד |
| − | | | + | |} |
|- | |- | ||
|} | |} | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | + | ::Think by how much the first 4 is less than 10, it is 6. | |
| − | + | ::<math>\scriptstyle{\color{blue}{10-4=6}}</math> | |
| − | + | |style="text-align:right;"|וחשוב כמה ד' ראשונה פחות מי' ויהיה ו‫' | |
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Think also by how much the second [4] is less than 10, you find it is also 6. |
| + | ::<math>\scriptstyle{\color{blue}{10-4=6}}</math> | ||
| + | |style="text-align:right;"|וחשוב גם השנייה כמה השנייה פחות מי' ותמצא ג"כ ו‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Multiply 6 by 6, the result is 36. |
| + | ::<math>\scriptstyle{\color{blue}{6\times6=36}}</math> | ||
| + | |style="text-align:right;"|ואז תכפול ו' על ו' ויעלה בידך ו'ג‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Think by how much the two 4 are less than ten, it is 2. |
| + | ::<math>\scriptstyle{\color{blue}{10-\left(4+4\right)=2}}</math> | ||
| + | |style="text-align:right;"|אז חשוב כמה השני ד' פחותי' מעשר' ויהיה ב‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Subtract 2 in the second rank from 3, you are left with 16 |
| + | |style="text-align:right;"|השלך ב' ממעלה שנייה מהג' וישאר לך ו'א‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::We find that 4 times 4 is 16. |
| + | ::<math>\scriptstyle{\color{blue}{4\times4=16}}</math> | ||
| + | |style="text-align:right;"|נמצא ד'פ'ד' הוא ו'א‫' | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | ::<span style=color:green>[Illustration of the procedure:]</span> | ||
| + | ::{| | ||
|- | |- | ||
| − | | | + | |<span style="color:purple">4</span>||rowspan="3"|<math>\scriptstyle\xrightarrow{\begin{cases}\scriptstyle{\color{Purple}{10-4}}={\color{blue}{6}}\\\scriptstyle{\color{Purple}{10-4}}={\color{blue}{6}}\end{cases}}</math>||[4]||rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{Purple}{6\sdot6}}={\color{blue}{36}}}</math>||[<span style="color:#0000FF>36</span>]||rowspan="3"|<math>\scriptstyle\xrightarrow{\begin{cases}\scriptstyle{\color{Purple}{10-\left(4+4\right)=2}}\\\scriptstyle{\color{Purple}{3-2}}={\color{blue}{1}}\end{cases}}</math>||<span style="color:#0000FF>1</span>6 |
| − | |||
|- | |- | ||
| − | | | + | |<span style="color:purple">4</span>|| [4]|| [4]|| |
| − | |||
|- | |- | ||
| + | | ||[<span style="color:#0000FF>66</span>]||[66]|| | ||
|} | |} | ||
| − | |||
|- | |- | ||
| | | | ||
| − | + | :The same for all; note well | |
| − | + | |style="text-align:right;"|וכן כולם וא"ק | |
| − | + | |- | |
| − | + | | colspan="2"| | |
| + | *<math>\scriptstyle{\color{OliveGreen}{x+y<10\quad x\sdot y=\left[\left(10-y\right)\sdot\left(10-x\right)\right]+\left[10\sdot\left(x+y\right)\right]-\left(10\sdot10\right)}}</math> | ||
| + | : | ||
|- | |- | ||
| − | | | + | |If the numbers that you want to multiply one by the other are less than 10, when you sum them together: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|‫<ref>MS Oxford 60, 151v, 161r: supplement</ref>ואם יהיה המספר אשר תרצה לכפול זע"ז פחות מי' כשתצרף שני המספרים יחד |
|- | |- | ||
| | | | ||
| − | * | + | :*{{#annot:4×3|156|wODz}}Example: if you want to multiply 4 by 3. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle4\times3</math> |
| + | |style="text-align:right;"|דימיון אם בקשת לכפול ד' על ג‫'{{#annotend:wODz}} | ||
|- | |- | ||
| | | | ||
| − | + | ::Take the difference of each from 10, i.e. from 4 to 10, it is 6 and from 3 to 10, it is 7 | |
| − | |style="text-align:right;"| | + | ::6 times 7 is 42. |
| + | ::<math>\scriptstyle{\color{blue}{\left(10-4\right)\times\left(10-3\right)=6\times7=42}}</math> | ||
| + | |style="text-align:right;"|אז תקח המרחק מן י' מכל אחד דהיינו מד' עד י' ו' ומג' עד י' ז‫'<br> | ||
| + | ופ"ז ב"ד | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | ::Write this 42 for remembrance. |
| + | |style="text-align:right;"|אותו ב"ד כתו' לזכרון<br> | ||
| + | [בד] | ||
|- | |- | ||
| | | | ||
| − | + | ::Sum 4 and 3 together, it is 7. | |
| − | + | ::<math>\scriptstyle{\color{blue}{4+3=7}}</math> | |
| − | + | |style="text-align:right;"|ואח"כ {{#annot:term|178,1810|GrJ7}}צרוף{{#annotend:GrJ7}} ד' ג' יחד ויהיה ז‫' | |
| − | |||
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | : | + | ::Write this 7 next to 42 to the left, like this: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אות ז' כתוב לצד שמאל אצל ב"ד כזה<br> |
| + | [בדז] | ||
|- | |- | ||
| | | | ||
| − | + | ::Subtract 10 from [the sum of] the numerals on the left, 12 remains, which is the number that you want to know. | |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\left(7+4\right)-10=1}}</math> |
| + | ::<math>\scriptstyle{\color{blue}{4\times3=12}}</math> | ||
| + | |style="text-align:right;"|ואח"כ השלך י' מצד אותיות של שמאל וישאר ב'א' היינו החשבון אשר בקשת לידע | ||
|- | |- | ||
| − | |< | + | | colspan="2"| |
| − | + | ::<span style=color:green>[Illustration of the procedure:]</span> | |
| + | ::{| | ||
|- | |- | ||
| − | | | + | |<math>\scriptstyle\xrightarrow{{\color{Purple}{\left(10-4\right)\sdot\left(10-3\right)=6\sdot7}}={\color{blue}{42}}}</math>||<span style="color:#0000FF>42</span>||<math>\scriptstyle\xrightarrow{{\color{Purple}{4+3}}={\color{blue}{7}}}</math>||<span style="color:#0000FF>7</span>42||<math>\scriptstyle\xrightarrow{{\color{Purple}{\left(7+4\right)-10}}={\color{blue}{1}}}</math>||<span style="color:#0000FF>1</span>2 |
| − | + | |} | |
| − | |||
| − | |||
| − | |style=" | ||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | + | :Deduce from this. | |
| − | + | |style="text-align:right;"|וכפי זה תקיש | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | :I wrote this even though it is an axiom for the calculation that you wanted, in order to inform you that in this way [...] | |
| − | + | |style="text-align:right;"|והנה כתבתי זה אף כי הוא מושכל ראשון החשבון אשר בקשת להודיעך שאין בדרך הזה [...]‫<ref>MS Oxford 60, 151v, 161r: end of the supplement</ref> | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| − | | | + | |I have found another method for the multiplication of units in another book: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|‫<ref>MS New York, 94r-v; MS Oxford 60, 161r; MS Oxford 440, 115r, 124r: supplement</ref>דרך אחרת לכפל קטן בספר אחר מצאתי |
|- | |- | ||
| − | | | + | | colspan="2"| |
| − | + | *<math>\scriptstyle{\color{OliveGreen}{x,y<10\quad x\sdot y=\left[\left(10-y\right)\sdot\left(10-x\right)\right]+\left[10\sdot\left[\left(x+y\right)-10\right]\right]}}</math> | |
| − | |||
|- | |- | ||
| − | | | + | |Or in this way: take the difference from 10 of each [of the units] that you want to multiply, multiply the two differences by each other and write the result for remembrance. |
| − | + | |style="text-align:right;"|או כלך בדרך זו<br> | |
| − | |style="text-align:right;"| | + | קח המרחק עד י’ מן כל אחד ואחד שתרצה לכפול יחד וחשוב אותו ב’ מרחקים זה על זה ומה שיעלה בידך כתוב לזכרון |
|- | |- | ||
| − | | | + | |Then, sum [the multipliers] together according to the chapter of addition, subtract 10 from the result, and write [the remainder] below to the left of what you have already wrote. |
| − | + | |style="text-align:right;"|אחר כך צורפם יחד בשער החיבור והעולה בידך קח מה שלמטה מן י’ וי’ השלך מידך וכתוב אותו למטה בצד שמאל למה שכתבת כבר | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| − | | | + | |Your calculation will be correct. |
| − | + | |style="text-align:right;"|ויהיה חשבונך מכוון | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*{{#annot:7×9|156|lzGK}}Example: you wish to multiply 7 times 9. |
| + | ::<math>\scriptstyle7\times9</math> | ||
| + | |style="text-align:right;"|דמיון הנה רצונך לכפול ז’ פעמים ט‫’{{#annotend:lzGK}} | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{ | + | ::Take the difference from 7 to 10, i.e. 3, and the difference from 9 to 10, i.e. 1, and say: 3 times 1 are 3. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\left(10-7\right)\times\left(10-9\right)=3\times1=3}}</math> |
| + | |style="text-align:right;"|קח המרחק מן הז' עד י' דהיינו ג' והמרחק מן ט’ עד י’ דהיינו א’ ואמור ג’ פעמים א’ היינו ג‫’ | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::Write this 3 for remembrance as this: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אותו ג’ כתוב לזכרון כזה<br> |
| + | ג | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{ | + | ::*Then, add 7 to 9, the result is 16, meaning sixteen, subtract the 10 and take the 6. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\left(7+9\right)-10=16-10=6}}</math> |
| + | |style="text-align:right;"|אחר כך חבור ז' על ט' ויעלה ו"א פי' י"ו השלך הי’ והו’ קח בידך | ||
|- | |- | ||
| | | | ||
| − | + | ::Write it to the left, next to the remaining 3, so it will be sixty three, like this: | |
| − | :<math>\scriptstyle\ | + | ::<math>\scriptstyle{\color{blue}{7\times9=63}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכתוב אותה בצד שמאל אצל הג’ הנשארת ויהיה כזה<br> |
| + | גו<br> | ||
| + | ששי' ושלש | ||
|- | |- | ||
| − | | | + | | colspan="2"| |
| − | + | ::<span style=color:green>[Illustration of the procedure:]</span> | |
| + | ::{| | ||
|- | |- | ||
| − | | | + | |<math>\scriptstyle\xrightarrow{{\color{Purple}{\left(10-7\right)\sdot\left(10-9\right)=3\sdot1}}={\color{blue}{3}}}</math>||<span style="color:#0000FF>3</span>||<math>\scriptstyle\xrightarrow{{\color{Purple}{\left(7+9\right)-10=16-10}}={\color{blue}{6}}}</math>||<span style="color:#0000FF>6</span>3 |
| − | + | |} | |
| − | |style=" | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Look carefully. |
| + | |style="text-align:right;"|ועיין | ||
|- | |- | ||
| | | | ||
| − | : | + | :*{{#annot:6×8|156|s2II}}Another example: you wish to multiply 6 times 8. |
| − | :<math>\ | + | ::<math>\scriptstyle6\times8</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|דמיון אחר הנה רצונך לכפול ו’ פעמים ח‫’{{#annotend:s2II}} |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Take the difference from 6 to 10, i.e. 4, and the difference from 8 [to 10], i.e. 2, and say: 2 times 4 are 8. |
| + | ::<math>\scriptstyle{\color{blue}{\left(10-6\right)\times\left(10-8\right)=4\times2=8}}</math> | ||
| + | |style="text-align:right;"|קח המרחק מן ו’ עד י’ דהיינו ד’ והמרחק מן ח’ היינו ב’ ואמור ב’ פעמים ד’ היינו ח‫’ | ||
|- | |- | ||
| | | | ||
| − | + | ::Write this 8 for remembrance as this: | |
| − | : | + | |style="text-align:right;"|אותו ח' כתוב לזכרון<br> |
| − | |style="text-align:right;"| | + | [ח] |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Then, add 6 to 8, the result is 14, subtract 10 and take 4. |
| + | ::<math>\scriptstyle{\color{blue}{\left(6+8\right)-10=14-10=4}}</math> | ||
| + | |style="text-align:right;"|אחר כך חבור ו' על ח' ויעלה י”ד השלך י' וקח ד' בידך | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle{\color{blue}{ | + | ::Write 4 to the left, next to the 8 that you have already wrote, the result is 48, i.e. 8 times 6. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{6\times8=48}}</math> |
| + | |style="text-align:right;"|וכתוב ד' בצד שמאל אל ח' אשר כתבת כבר ויעלה מ”ח היינו חשבון ח'פ'ו‫'<br> | ||
| + | [חד] | ||
|- | |- | ||
| − | | | + | | colspan="2"| |
| − | + | ::<span style=color:green>[Illustration of the procedure:]</span> | |
| + | ::{| | ||
|- | |- | ||
| − | | | + | |<math>\scriptstyle\xrightarrow{{\color{Purple}{\left(10-6\right)\sdot\left(10-8\right)=4\sdot2}}={\color{blue}{8}}}</math>||[<span style="color:#0000FF>8</span>]||<math>\scriptstyle\xrightarrow{{\color{Purple}{\left(6+8\right)-10=14-10}}={\color{blue}{4}}}</math>||[<span style="color:#0000FF>4</span>8] |
| − | + | |} | |
| − | :<math>\scriptstyle\ | ||
| − | |style=" | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Look carefully. |
| + | |style="text-align:right;"|עיין ודוק‫<ref>end of the supplement</ref> | ||
|- | |- | ||
| | | | ||
| − | : | + | :*{{#annot:5×6|156|XdEP}}Another example: if you want to know 5 times 6. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle5\times6</math> |
| + | |style="text-align:right;"|‫<ref>MS Oxford 440, 115r: supplement</ref>דמיון אחר אם תרצה לידע הפ"ו{{#annotend:XdEP}} | ||
|- | |- | ||
| | | | ||
| − | : | + | ::Take the difference from 5 to 10, it is 5. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\left(10-5\right){\color{Purple}{\times\left(10-6\right)}}=5{\color{Purple}{\times4=20}}}}</math> |
| + | |style="text-align:right;"|קח המרחק מן ה' עד י' ויהיה ה‫' | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{ | + | ::Sum 5 and 6 together, it is 1[1], subtract ten and you are left with 1. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\left(5+6\right)-10=1{\color{Purple}{1}}-10=1}}</math> |
| + | |style="text-align:right;"|צרפם יחד הה’ והו’ ויהיה א' השלך עשרה ונשאר לך א‫' | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\ | + | ::Add it to the [2] and it is 3, so we find it 30. Note well. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{5\times6=30}}</math> |
| + | |style="text-align:right;"|צרפם 0"ב ויהיה ג' נמצא ל' ודוק‫<ref>MS Oxford 440, 115r: end of the supplement</ref> | ||
|- | |- | ||
| − | | | + | | colspan="2"| |
| − | ::< | + | ::<span style=color:green>[Illustration of the procedure:]</span> |
| − | + | ::{| | |
|- | |- | ||
| − | | | + | |<math>\scriptstyle\xrightarrow{{\color{Purple}{\left(10-5\right)\sdot\left(10-6\right)=5\sdot4}}={\color{blue}{20}}}</math>||[<span style="color:#0000FF>20</span>]||<math>\scriptstyle\xrightarrow{\begin{cases}\scriptstyle{\color{Purple}{\left(5+6\right)-10=1}}\\\scriptstyle{\color{Purple}{2+1}}={\color{blue}{3}}\end{cases}}</math>||[<span style="color:#0000FF>3</span>0] |
| − | + | |} | |
| − | |||
| − | |||
| − | |style=" | ||
|- | |- | ||
| + | !<span style=color:green>Multiplication Table</span> | ||
| | | | ||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | *<span style=color:green>multiplication table of 1 to 9</span> |
| − | |||
| − | |||
| | | | ||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |I will write to you a precise table of multiplication of units, in order that you will be able to observe easily all that you want without any calculation, from 1 times 1 to 9 times 9. Note well. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והנה ארשום לך לוח מתוקנת על שער הכפל קטן כדי שתוכל לראות בקלות כל מה שתרצה בלא שום חשבון מן א' פעמי' א' עד ט' פעמים ט' ודו"ק |
|- | |- | ||
| | | | ||
| − | : | + | *<span style=color:green>half multiplication table [MS Oxford 440, 115r; Oxford 60, 175r]</span> |
| − | |||
| − | |||
| | | | ||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |I made a half table in order to find every number, since you do not need that much. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והחצי לוח עשיתי למצוא כל חשבון ואינך צריך הרבה כל כך |
|- | |- | ||
| − | | | + | |The chapter on the multiplication of units is completed. |
| − | + | |style="text-align:right;"|סליק שער כפל קטן | |
| − | |||
| − | |style="text-align:right;"| | ||
|- | |- | ||
| − | | | + | |You shall become wise in all that you do. |
| − | + | |style="text-align:right;"|ואז תשכיל כל אשר תעשה | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | : | + | |
| − | |style="text-align:right;"| | + | == Chapter Two: Addition == |
| + | |||
| + | |style="width:45%; text-align:right;"|‫<ref>110v</ref><big>שער ה{{#annot:term|154,1208|yGi5}}חיבור{{#annotend:yGi5}}</big> | ||
|- | |- | ||
| | | | ||
| − | : | + | *{{#annot:3372+3392|154|5lLo}}If you wish to sum three thousand three hundred and seventy-two with three thousand three hundred ninety-two. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle3372+3392</math> |
| + | |style="text-align:right;"|אם תרצה לחבר שנים ושבעים וג' מאות וג' אלפים עם שנים ותשעים וג' מאות וג' אלפי‫'{{#annotend:5lLo}} | ||
|- | |- | ||
| | | | ||
| − | + | ::Write as follows: | |
| − | : | + | |style="text-align:right;"|תכתוב כך |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | : | + | ::{|class="wikitable" style="color:blue; text-align:center;" |
| − | |||
|- | |- | ||
| | | | ||
| − | + | {|style="margin-left: auto; margin-right: 0px;" | |
| − | |style=" | ||
|- | |- | ||
| − | | | + | |3||3||7||2 |
| − | |||
| − | |||
| − | | | ||
|- | |- | ||
| − | | | + | |3||3||9||2 |
| − | + | |} | |
| − | | | ||
|- | |- | ||
| + | |} | ||
| | | | ||
| − | + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | |
| − | |||
|- | |- | ||
| | | | ||
| − | + | {|style="margin-left: auto; margin-right: 0px;" | |
| − | |style=" | ||
|- | |- | ||
| − | | | + | |ג||ג||ז||ב |
| − | |||
| − | | | ||
|- | |- | ||
| − | | | + | |ג||ג||ט||ב |
| − | + | |} | |
| − | | | ||
|- | |- | ||
| − | | | + | |} |
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Add the bottom 2 to the upper 2; it is 4. |
| + | ::<math>\scriptstyle{\color{blue}{2+2=4}}</math> | ||
| + | |style="text-align:right;"|{{#annot:term|178,1165|W4ax}}תחבר{{#annotend:W4ax}} ב' התחתונה על ב' העליונה ויהיה ד‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | ::{|class="wikitable" style="color:blue; text-align:center;" |
| − | |||
|- | |- | ||
| | | | ||
| − | + | {|style="margin-left: auto; margin-right: 0px;" | |
| − | |style=" | ||
|- | |- | ||
| − | | | + | |3||3||7||2 |
| − | |||
| − | | | ||
|- | |- | ||
| − | | | + | |3||3||9||2 |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | | | ||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | | || || ||4 |
| − | + | |} | |
| − | | | ||
|- | |- | ||
| + | |} | ||
| | | | ||
| − | + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | |
| − | |style="text-align: | ||
|- | |- | ||
| | | | ||
| − | + | {|style="margin-left: auto; margin-right: 0px;" | |
| − | |||
| − | |||
| − | |style=" | ||
|- | |- | ||
| − | | | + | |ג||ג||ז||ב |
| − | |||
| − | | | ||
|- | |- | ||
| − | | | + | |ג||ג||ט||ב |
| − | |||
| − | | | ||
|- | |- | ||
| − | | | + | | || || ||ד |
| − | + | |} | |
| − | | | ||
|- | |- | ||
| − | | | + | |} |
| − | + | ||
| − | |||
|- | |- | ||
| | | | ||
| − | :: | + | ::Add the bottom 9 to the upper 7; it is 16. |
| − | + | ::<math>\scriptstyle{\color{blue}{9+7=16}}</math> | |
| − | + | |style="text-align:right;"|תחבר ט' התחתונה עם הז' ויהיה ו"א | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | : | + | ::Write the 6 in the second rank, after the 4 that you wrote, like this: |
| − | + | |style="text-align:right;"|הו' תכתוב במעלה השנייה אחר הד' [שכתבת כזה‫]‫<ref>M om.</ref> | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::{|class="wikitable" style="color:blue; text-align:center;" |
| − | |||
| − | : | ||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | + | {|style="margin-left: auto; margin-right: 0px;" | |
| − | |||
| − | |||
| − | |||
| − | |style=" | ||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |6||4 |
| − | + | |} | |
| − | |||
| − | |||
| − | | | ||
| − | |||
| − | |||
|- | |- | ||
| + | |} | ||
| | | | ||
| − | + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | |
| − | |||
|- | |- | ||
| | | | ||
| − | |style=" | + | {|style="margin-left: auto; margin-right: 0px;" |
|- | |- | ||
| − | | | + | |ו||ד |
| − | + | |} | |
| − | + | |- | |
| − | + | |} | |
| − | | | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::Add the remaining 1 to the threes that follow it in the third rank, like this: |
| − | : | + | |style="text-align:right;"|והא' [הנשארת]‫<ref>M om.</ref> תחבר לג'ג' הראשונה שהם זו למעלה מזו כזה במעלה השלישית |
| − | |||
| − | |||
| − | |style="text-align:right;"| | ||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | :: | + | ::{|class="wikitable" style="color:blue; text-align:center;" |
| − | |||
| − | |||
| − | : | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | + | {|style="margin-left: auto; margin-right: 0px;" | |
| − | : | ||
| − | |||
| − | |||
| − | : | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |3 |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |3 |
| − | | | + | |} |
|- | |- | ||
| + | |} | ||
| | | | ||
| − | + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | |
| − | |||
|- | |- | ||
| | | | ||
| − | + | {|style="margin-left: auto; margin-right: 0px;" | |
| − | |style=" | ||
|- | |- | ||
| − | | | + | |ג |
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |ג |
| − | + | |} | |
| − | |||
|- | |- | ||
| − | | | + | |} |
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | :::<math>\scriptstyle{\color{blue}{ | + | ::So, the total is 7 in the third rank, like this: |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{1+3+3=7}}</math>: |
| + | |style="text-align:right;"|ויהיה הכל ז' במעלה השלישי' כזה | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::{|class="wikitable" style="color:blue; text-align:center;" |
| − | |||
|- | |- | ||
| | | | ||
| − | + | {|style="margin-left: auto; margin-right: 0px;" | |
| − | |style=" | ||
|- | |- | ||
| − | | | + | |7||6||4 |
| − | + | |} | |
| − | |||
|- | |- | ||
| + | |} | ||
| | | | ||
| − | + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | |
| − | |||
|- | |- | ||
| | | | ||
| − | + | {|style="margin-left: auto; margin-right: 0px;" | |
| − | |style=" | ||
|- | |- | ||
| − | | | + | |ז||ו||ד |
| − | + | |} | |
| − | | | ||
|- | |- | ||
| − | | | + | |} |
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{ | + | ::Then, add the two last threes in the fourth rank; their sum is 6. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{3+3=6}}</math> |
| + | ::Write this 6 in the fourth rank, after 764 that you wrote, like this: | ||
| + | |style="text-align:right;"|ואח"כ תחבר הג'ג' האחרוני' [במעלה הרביעי']‫<ref>M om.</ref> [ויהיה חבורם]‫<ref>M והם</ref> ו' [אות' ו' כתוב במעלה הרביעי' אחר דוז שכתבת]‫<ref>M om.</ref> ויהיה כזה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align: | + | ::{|class="wikitable" style="color:blue; text-align:center;" |
|- | |- | ||
| | | | ||
| − | + | {|style="margin-left: auto; margin-right: 0px;" | |
| − | |||
| − | |style=" | ||
|- | |- | ||
| − | | | + | |6||7||6||4 |
| − | | | + | |} |
|- | |- | ||
| + | |} | ||
| | | | ||
| − | + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | |
| − | :: | ||
| − | |||
|- | |- | ||
| | | | ||
| − | + | {|style="margin-left: auto; margin-right: 0px;" | |
| − | |style=" | ||
|- | |- | ||
| − | | | + | |ו||ז||ו||ד |
| − | + | |} | |
| − | | | ||
|- | |- | ||
| − | | | + | |} |
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::The procedure is complete and the sum is six thousand seven hundred and sixty-four, i.e. as follows: |
| + | |style="text-align:right;"|‫[ותשלם המלאכה ויהיה {{#annot:term|388,1208|fuG0}}חבורו{{#annotend:fuG0}} ארבע וששי' ז' מאות ו' אלפי' דהיינו כזה]‫<ref>M om.</ref> | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::{|class="wikitable" style="color:blue; text-align:center;" |
| − | |||
|- | |- | ||
| | | | ||
| − | + | {|style="margin-left: auto; margin-right: 0px;" | |
| − | |style=" | ||
|- | |- | ||
| − | | | + | |6||7||6||4 |
| − | | | + | |} |
|- | |- | ||
| + | |} | ||
| | | | ||
| − | + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | |
| − | |||
|- | |- | ||
| | | | ||
| − | + | {|style="margin-left: auto; margin-right: 0px;" | |
| − | |style=" | ||
|- | |- | ||
| − | | | + | |ו||ז||ו||ד |
| − | | | + | |} |
|- | |- | ||
| − | | | + | |} |
| − | |||
|- | |- | ||
| − | | | + | | colspan="2"| |
| − | + | ::<span style=color:green>[Illustration of the procedure:]</span> | |
| − | : | + | ::{| |
| − | |||
| − | : | ||
| − | : | ||
| − | |||
| − | |||
| − | | | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |337<span style="color:purple">2</span>||rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{Purple}{2+2}}={\color{blue}{4}}}</math>||[33<span style="color:purple">7</span>2]||rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{Purple}{7+9}}={\color{green}{1}}{\color{blue}{6}}}</math>||[3<span style="color:purple">3</span>72]||rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{Purple}{{\color{green}{1}}+3+3}}={\color{blue}{7}}}</math>||[<span style="color:purple">3</span>372]||rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{Purple}{3+3}}={\color{blue}{6}}}</math>||[3372] |
| − | ::<math>\scriptstyle{\color{blue}{6+ | ||
| − | |style=" | ||
|- | |- | ||
| − | | | + | |339<span style="color:purple">2</span>||[33<span style="color:purple">9</span>2]||[3<span style="color:purple">3</span>92]||[<span style="color:purple">3</span>392]||[3392] |
| − | ::< | ||
| − | |style=" | ||
|- | |- | ||
| − | | | + | | ||   <span style="color:#0000FF>4</span>||   <span style="color:#0000FF>6</span>4||  <span style="color:#0000FF>7</span>64|| <span style="color:#0000FF>6</span>764 |
| − | :< | + | |} |
| − | |style=" | ||
|- | |- | ||
| − | | | + | |The highest rank of the sum exceeds the addend by one rank to the left at most, sometimes it does not exceed [its ranks] at all. |
| − | + | |style="text-align:right;"|לעולם לא תמצא הטור מן החיבור אשר בעליונה עוברת על ה{{#annot:term|787,1220|daNJ}}מחובר{{#annotend:daNJ}} רק מעלה לשמאל לכל היותר ולפעמים לא תעבור כלל ול"ד | |
| − | |||
| − | |style="text-align:right;"| | ||
|- | |- | ||
| − | | | + | |Here follows a chapter on addition, to add every thing and to establish its methods |
| − | + | |style="text-align:right;"|‫<ref>MS Paris 1088, 7v</ref>הילך שער חיבור לחבר כל דבר [..] ולישב על אופניו | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| − | | | + | |Such as, if you wish to sum some numbers together. |
| − | + | |style="text-align:right;"|כגון אם תרצה לחבר כמה חשבונות יחד | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| − | | | + | |Now, I sum one number and according to this you shall apply for others: |
| − | + | |style="text-align:right;"|ועתה אחבר חשבון אחד ומהם תבין אחר | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| − | | | + | |Write numeral by numeral [lit. letter by letter], but not as is written in the multiplication chapter, but write as I will demonstrate, with God's help. |
| − | + | |style="text-align:right;"|ותכתו' אות תחת אות ולא תכתו' כאשר כותבין בשער כפל אלא תכתו' כזה שאראך בע"ה | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | ::<math>\ | + | *{{#annot:3372+9892|154|ZOaG}}Example: we want to sum 3372 with 9892. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle3372+9892</math> |
| + | |style="text-align:right;"|דומיון בקשנו לחבר ע"ב וג' מאות וג' אלפי' עם צ"ב וח' מאות וט' אלפים{{#annotend:ZOaG}} | ||
|- | |- | ||
| | | | ||
| − | : | + | ::Here is how you write it and its diagram is as follows: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|הילך היאך תכתוב אותו וצורתו כזה |
|- | |- | ||
| | | | ||
| − | + | ::{|class="wikitable" style="color:blue; text-align:center;" | |
| − | : | ||
| − | |||
|- | |- | ||
| | | | ||
| − | + | {|style="margin-left: auto; margin-right: 0px;" | |
| − | |style=" | ||
|- | |- | ||
| − | | | + | |3||3||7||2 |
| − | |||
| − | | | ||
|- | |- | ||
| − | | | + | |9||8||9||2 |
| − | + | |} | |
| − | |||
|- | |- | ||
| + | |} | ||
| | | | ||
| − | + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | |
| − | |||
|- | |- | ||
| | | | ||
| − | + | {|style="margin-left: auto; margin-right: 0px;" | |
| − | |style=" | ||
|- | |- | ||
| − | | | + | |ג||ג||ז||ב |
| − | |||
| − | | | ||
|- | |- | ||
| − | | | + | |ט||ח||ט||ב |
| − | + | |} | |
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |} |
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | ::We add the bottom 2 to the upper 2; it is 4. |
| − | :: | + | ::<math>\scriptstyle{\color{blue}{2+2=4}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|נחבר ב' תחתונ' עם ב' עליונ' הרי ד‫' |
|- | |- | ||
| | | | ||
| − | + | ::We write the 4 corresponding to the bottom 2. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אות' ד' נכתוב נגד ב' תחתונ‫' |
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle\ | + | ::We add also the bottom 9 to the upper 7; the result is 16. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{9+7=16}}</math> |
| − | + | |style="text-align:right;"|נחבר כמו כן ט' תחתונ' עם ז' עליונ' ויעלה ו"א | |
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::We write 6 beneath the 9. |
| + | |style="text-align:right;"|נכתוב ו' תחת הט‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | ::We add 1 to the next digit in the top row: there is a 3 there. We add 1 to it; it is as if we write 4 in the place of the 3. |
| − | :<math>\scriptstyle\ | + | ::<math>\scriptstyle{\color{blue}{1+3=4}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ו{{#annot:term|178,1206|PctD}}נוסיף{{#annotend:PctD}} א' על אות שבשיט' עליונ' הסמוכ' לה ושם יש ג' ונוסיף א' עליה ויהיה כאילו נכתו' ד' במקום ג‫' |
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | + | ::We add the upper 4 to the bottom 8; the result is 12. | |
| − | :<math>\scriptstyle\ | + | ::<math>\scriptstyle{\color{blue}{4+8=12}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ונחבר ד' עליונ' עם ח' תחתונ' ויעלה ב"א |
|- | |- | ||
| | | | ||
| − | :: | + | ::We write 2 beneath the 8. |
| − | + | |style="text-align:right;"|נכתו' ב' למטה תחת הח‫' | |
| − | |style="text-align:right;"| | ||
| − | |||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle\ | + | ::We add 1 to the digit next to the upper 4: there is a 3 there. We add 1 to it; it is 4. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{1+3=4}}</math> |
| + | |style="text-align:right;"|ונוסיף א' על אות הסמוך לד' עליונ' ושם יש ג' ונוסיף א' עליה ויהא ד‫' | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle{\color{blue}{ | + | ::We add the upper 4 to the bottom 9; the result is 13. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{4+9=13}}</math> |
| + | |style="text-align:right;"|נחבר ד' עליונ' על ט' שלמטה ויעלה ג"א | ||
|- | |- | ||
| | | | ||
| − | + | ::We write 3 beneath the 9 and 1 after it; it is like this: | |
| − | : | + | |style="text-align:right;"|נכתוב ג' למט' תחת הט' וא' נכתוב לאחריה ויהיה כזה |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | : | + | ::{|class="wikitable" style="color:blue; text-align:center;" |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | + | {|style="margin-left: auto; margin-right: 0px;" | |
| − | |||
| − | |style=" | ||
|- | |- | ||
| − | | | + | | ||3||3||7||2 |
| − | |||
| − | | | ||
|- | |- | ||
| − | | | + | | ||9||8||9||2 |
| − | |||
| − | |||
| − | |||
| − | | | ||
|- | |- | ||
| − | | | + | |1||3||2||6||4 |
| − | + | |} | |
| − | |||
| − | |||
| − | |||
|- | |- | ||
| + | |} | ||
| | | | ||
| − | + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | |
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | + | {|style="margin-left: auto; margin-right: 0px;" | |
| − | |style=" | ||
|- | |- | ||
| − | | | + | | ||ג||ג||ז||ב |
| − | |||
| − | | | ||
|- | |- | ||
| − | | | + | | ||ט||ח||ט||ב |
| − | |||
| − | | | ||
|- | |- | ||
| − | | | + | |א||ג||ב||ו||ד |
| − | + | |} | |
| − | |||
| − | |||
| − | | | ||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |} |
| − | |||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |Do not forget this: when the 1 remains from the first rank, I add it to the rank after it. Since the 1 I have left is also as tenths of the first rank, so I add it to it frequently. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|זה הכלל לא ימוש מפיך כשמותר הא' משיט' ראשונ' אני מוסיף אות' על שיט' שלאחריה<br> |
| + | כי הא' הנותרת לי היא כמו כן מן העשיריות נגד שיט' ראשונ' לכך אני מוסיף אות' תדיר עליה | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::We find that the result of the above addition is 13 thousand, two hundred and 64. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|נמצא החיבור שחברנו לעיל הוא עולה ס"ד ומאתים וי"ג אלפים ודוק |
|- | |- | ||
| − | | | + | | colspan="2"| |
| − | :: | + | ::<span style=color:green>[Illustration of the procedure:]</span> |
| − | + | ::{| | |
|- | |- | ||
| − | | | + | |337<span style="color:purple>2</span>||rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{Purple}{2+2}}={\color{blue}{4}}}</math>||33<span style="color:purple">7</span>2||rowspan="3"|<math>\scriptstyle\xrightarrow{\begin{cases}\scriptstyle{\color{Purple}{7+9}}={\color{green}{1}}{\color{blue}{6}}\\\scriptstyle{\color{Purple}{3+}}{\color{green}{1}}={\color{blue}{4}}\end{cases}}</math>||3<span style="color:#0000FF>4</span>72||rowspan="3"|<math>\scriptstyle\xrightarrow{\begin{cases}\scriptstyle{\color{Purple}{4+8}}={\color{green}{1}}{\color{blue}{2}}\\\scriptstyle{\color{Purple}{3+}}{\color{green}{1}}={\color{blue}{4}}\end{cases}}</math>||<span style="color:#0000FF>4</span>472||rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{Purple}{4+9}}={\color{blue}{13}}}</math>|| 3372 |
| − | ::<math>\scriptstyle{\color{blue}{ | ||
| − | |style=" | ||
|- | |- | ||
| − | | | + | |989<span style="color:purple">2</span>||98<span style="color:purple">9</span>2||9<span style="color:purple">8</span>92||<span style="color:purple">9</span>892|| 9892 |
| − | |||
| − | |style=" | ||
|- | |- | ||
| − | | | + | | ||   <span style="color:#0000FF>4</span>||  <span style="color:#0000FF>6</span>4|| <span style="color:#0000FF>2</span>64||<span style="color:#0000FF>13</span>264 |
| − | : | + | |} |
| − | |style=" | ||
|- | |- | ||
| − | | | + | |This diagram is from Latin and is called "addirin" [= addition]. |
| − | + | |style="text-align:right;"|‫<ref>MS Paris158, 198v</ref>ציור זה בגלחת ונקרא {{#annot:term|154|89Mu}}אדירין{{#annotend:89Mu}} | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| − | | | + | | colspan="2"| |
| − | |style="text-align:right;" | + | ::{|class="wikitable" style="color: blue; text-align:right;" |
|- | |- | ||
| − | | | + | |<math>\scriptstyle{\color{blue}{78312+87547}}</math>||<math>\scriptstyle{\color{blue}{68975+87496}}</math>||<math>\scriptstyle{\color{blue}{37064+52056}}</math> |
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | |style=" | + | {|style="float:right;" |
|- | |- | ||
| − | + | |style="text-align:right;"| ||7||8||3||1||2 | |
| − | |||
| − | |style="text-align:right;"| | ||
|- | |- | ||
| + | |style="text-align:right;"| ||8||7||5||4||7 | ||
| + | |} | ||
| | | | ||
| − | + | {|style="float:right;" | |
| − | |style=" | ||
|- | |- | ||
| − | + | |style="text-align:right;"| ||6||8||9||7||5 | |
| − | |||
| − | |style="text-align:right;"| | ||
|- | |- | ||
| + | |style="text-align:right;"| ||8||7||4||9||6 | ||
| + | |} | ||
| | | | ||
| − | + | {|style="float:right;" | |
| − | |style=" | ||
|- | |- | ||
| − | + | |style="text-align:right;"|3||7||0||6||4 | |
| − | |||
| − | |style="text-align:right;"| | ||
|- | |- | ||
| − | + | |style="text-align:right;"|5||2||0||5||6 | |
| − | + | |} | |
| − | |style="text-align:right;"| | + | |
|- | |- | ||
| | | | ||
| − | + | {|style="float:right;" | |
| − | |style=" | ||
|- | |- | ||
| + | |style="text-align:right;"|1||6||5||8||5||9 | ||
| + | |} | ||
| | | | ||
| − | + | {|style="float:right;" | |
| − | |||
| − | |style=" | ||
|- | |- | ||
| + | |style="text-align:right;"|1||5||6||4||7||1 | ||
| + | |} | ||
| | | | ||
| − | + | {|style="float:right;" | |
| − | |style=" | ||
|- | |- | ||
| − | + | |style="text-align:right;"|8||9||1||2||0 | |
| − | + | |} | |
| − | |style="text-align:right;"| | + | |} |
|- | |- | ||
| − | | | + | | colspan="2"| |
| − | + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | |
| − | |style="text-align: | ||
|- | |- | ||
| | | | ||
| − | | | + | {| |
|- | |- | ||
| − | + | |style="text-align:right;"| ||ז||ח||ג||א||ב | |
| − | |||
| − | |||
| − | |style="text-align:right;"| | ||
|- | |- | ||
| + | |style="text-align:right;"| ||ח||ז||ה||ד||ז | ||
| + | |} | ||
| | | | ||
| − | + | {| | |
| − | |||
|- | |- | ||
| − | + | |style="text-align:right;"| ||ו||ח||ט||ז||ה | |
| − | |||
| − | |style="text-align:right;"| | ||
|- | |- | ||
| + | |style="text-align:right;"| ||ח||ז||ד||ט||ו | ||
| + | |} | ||
| | | | ||
| − | + | {| | |
| − | |||
|- | |- | ||
| − | | | + | |style="text-align:right;"|ג||ז||0||ו||ד |
| − | : | + | |- |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ה||ב||0||ה||ו |
| + | |} | ||
| + | |||
| + | |- | ||
| + | | | ||
| + | {| | ||
| + | |- | ||
| + | |style="text-align:right;"|א||ו||ה||ח||ה||ט | ||
| + | |} | ||
| + | | | ||
| + | {| | ||
| + | |- | ||
| + | |style="text-align:right;"|א||ה||ו||ד||ז||א | ||
| + | |} | ||
| + | | | ||
| + | {| | ||
| + | |- | ||
| + | |style="text-align:right;"|ח||ט||א||ב||0 | ||
| + | |} | ||
| + | |} | ||
| + | |||
| + | |- | ||
| + | | | ||
| + | === <span style=color:green>Checking Methods</span> === | ||
| + | | | ||
| + | |- | ||
| + | |Now, know that I shall write you two checking method [lit. scales]: | ||
| + | |style="text-align:right;"|‫<ref>MS Paris158, 198v</ref>ועתה תדע שאכתוב לך שני {{#annot:term|354,1451|LdXM}}משקלים{{#annotend:LdXM}} | ||
| + | |- | ||
| + | | | ||
| + | :<span style=color:green>1) Casting out 9 by 9:</span> | ||
| + | :The first: cast out the nines as many as you can from the two upper lines and take what remains that is less that 9 as the weights of the scales. | ||
| + | |style="text-align:right;"|הא' {{#annot:term|1560,1265|9i9c}}השלך ט"ט{{#annotend:9i9c}} כל כמה שתוכל מב' שיטות עליונות ומה שנשמר לך למטה מט' קח בידך לאבני המשקל | ||
| + | |- | ||
| + | | | ||
| + | :Then, cast out the nines in the bottom line also, and if the scales of what remains is as the first scales, know that your calculation is correct. | ||
| + | |style="text-align:right;"|ואח"כ השלך ג"כ השיטה התחתונה בט"ט ומה שנשאר לך אם משקלו כאבן הראשון דע שכוינת חשבונך | ||
| + | |- | ||
| + | | | ||
| + | :<span style=color:green>2) Subtraction</span> | ||
| + | :The second scale is that one subtracts the two upper lines from the bottom line, and if all is gone as required no more and no less, then your calculation is correct. | ||
| + | |style="text-align:right;"|{{#annot:term|354,1451|mImR}}משקל{{#annotend:mImR}} השני יקח השני שיטות העליונות מהשיטה התחתונה אם יצא במבוקש בלי חסרון ויתרון אז חשבונך מכוון | ||
| + | |- | ||
| + | |Examine and you will find [that it is true]. | ||
| + | |style="text-align:right;"|ודוק ותמצא | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | == Chapter Three: Subtraction == | ||
| + | |||
| + | |style="width:45%; text-align:right;"|<big>שער ה{{#annot:term|155,1657|Otja}}חיסור{{#annotend:Otja}}</big> | ||
| + | |- | ||
| + | |If you want to subtract and deduct a number from a number, here is how you do that: | ||
| + | |style="width:45%; text-align:right;"|אם באת לחסיר ולנכות חשבון מתוך חשבון הילך איך לעשות | ||
| + | |- | ||
| + | |Write the number from which you wish to subtract and deduct above, according to its ranks, and write the number you subtract from it beneath, according to its ranks. | ||
| + | |style="text-align:right;"|תכתוב החשבון שאתה רוצה לחסיר ולנכות ממנו אותו חשבון תכתוב למעלה והחשבון שאתה מנכה תכתוב למטה כפי מעלותיו | ||
| + | |- | ||
| + | |Then subtract it from the number above. | ||
| + | |style="text-align:right;"|ואח"כ {{#annot:term|181,2201|poHT}}תנכה מן{{#annotend:poHT}} החשבון שלמעלה | ||
| + | |- | ||
| + | |Now, I shall write you an example to teach you: | ||
| + | |style="text-align:right;"|ועתה אכתוב לך הדמיון להשכילך | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:1000-999|155|F3VH}}We wish to subtract 9 hundred and ninety-nine from one thousand | ||
| + | :<math>\scriptstyle1000-999</math> | ||
| + | |style="text-align:right;"|הנה בקשנו לחסיר ט' מאות ותשעים ותשע מאלף{{#annotend:F3VH}} | ||
| + | |- | ||
| + | | | ||
| + | ::Here is how you write it: | ||
| + | |style="text-align:right;"|הילך היאך תכתוב אותו כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |1||0||0||0 | ||
| + | |- | ||
| + | | ||9||9||9 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |א||0||0||0 | ||
| + | |- | ||
| + | | ||ט||ט||ט | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::We find that the 1 that is in the upper line, in the fourth rank, is ten for the 9 that is third in the bottom line. | ||
| + | |style="text-align:right;"|נמצא הא' שבשיטה העליונה במעלה רביעי' עולה עשרה לגבי [ט']‫<ref>M om.</ref> שלישי' שבתחתונה | ||
| + | |- | ||
| + | | | ||
| + | ::Now, we subtract 9 from this ten; you are left with 1. | ||
| + | |style="text-align:right;"|עתה נחסיר מאותו עשרה ט' וישאר [לך]‫<ref>M om.</ref> א‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Write the 1 in the place of the third zero corresponding to the 9 that you have subtracted and erase the 9, like this: | ||
| + | |style="text-align:right;"|אותה א' תכתוב במקום גלגל השלישי נגד הט' שחסרת ואותו [ט'] ‫<ref>M: א'</ref> תמחוק כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |1||0||0 | ||
| + | |- | ||
| + | | ||9||9 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |א||0||0 | ||
| + | |- | ||
| + | | ||ט||ט | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Now, the 1 in the third rank of the upper line is also ten for the 9 in the bottom line. | ||
| + | |style="text-align:right;"|עכשיו כמו כן הא' שבמעלה שלישי' שבשיטה העליונה עולה י' לגבי ט' שבשיט' תחתונה | ||
| + | |- | ||
| + | | | ||
| + | ::We subtract the second 9; 1 remains. | ||
| + | |style="text-align:right;"|מאותו {{#annot:term|181,1362|Dk5a}}נחסיר{{#annotend:Dk5a}} ט' שנייה ונשאר א‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Write the 1 in the place of the second zero and erase the second 9; erase also the upper 1 that is in the third rank, from which we subtracted. It will be as follows: | ||
| + | |style="text-align:right;"|אות' א' תכתוב במקום הגלגל שני ותמחק ט' שנייה שחסרנו וגם תמחוק הא' העליונ' שבמעלה שלישי' שחסרנו ממנו ויהיה כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |1||0 | ||
| + | |- | ||
| + | | ||9 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |א||0 | ||
| + | |- | ||
| + | | ||ט | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Again, we subtract 9 that is beneath the zero from 1 that is above in the second rank, since it is also ten for the nine as above. | ||
| + | |style="text-align:right;"|שוב נחסיר ט' תחת הגלגל אמ' שלמעלה במעלה שנייה כי גם היא עולה י' לגבי ט' כדלעיל | ||
| + | |- | ||
| + | | | ||
| + | ::So, when we subtract 9 from 10, 1 remains. | ||
| + | |style="text-align:right;"|והנה כשנחסיר ט' מי' וישאר א‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Write the 1 in the place of the zero and erase the upper 1 that is in the second rank; erase also the 9. | ||
| + | |style="text-align:right;"|אות' א' תכתוב במקום הגלגל ונמחוק הא' העליונה אשר במעלה שנייה וגם הט' נמחק | ||
| + | |- | ||
| + | | | ||
| + | ::Then only one remains. | ||
| + | |style="text-align:right;"|ואז נשאר רק א‫' | ||
| + | |- | ||
| + | | | ||
| + | ::We find that when we subtract 999 from one thousand, 1 remains. | ||
| + | |style="text-align:right;"|נמצא כשחסירנו ט' מאות וצ"ט מאלף נשאר א‫' | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | ::{| | ||
| + | |- | ||
| + | |<span style="color:purple">10</span>00||rowspan="2"|<math>\scriptstyle\xrightarrow{{\color{Purple}{10-9}}={\color{blue}{1}}}</math>||<span style="color:#0000FF>1</span><span style="color:purple">0</span>0||rowspan="2"|<math>\scriptstyle\xrightarrow{{\color{Purple}{10-9}}={\color{blue}{1}}}</math>||<span style="color:#0000FF>1</span><span style="color:purple">0</span>||rowspan="2"|<math>\scriptstyle\xrightarrow{{\color{Purple}{10-9}}={\color{blue}{1}}}</math>||<span style="color:#0000FF>1</span> | ||
| + | |- | ||
| + | | <span style="color:purple">9</span>99|| <span style="color:purple">9</span>9|| <span style="color:purple">9</span>|| | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:654-321|155|j8ha}}Another example: if we wish to subtract 321 from 654. | ||
| + | :<math>\scriptstyle654-321</math> | ||
| + | |style="text-align:right;"|דומיון [אחר אם]‫<ref>M om.</ref> בקשנו לחסיר אב"ג מדה"ו{{#annotend:j8ha}} | ||
| + | |- | ||
| + | | | ||
| + | ::Write as follows: | ||
| + | |style="text-align:right;"|כתוב כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |6||5||4 | ||
| + | |- | ||
| + | |3||2||1 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ו||ה||ד | ||
| + | |- | ||
| + | |ג||ב||א | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::We subtract the bottom 3 from the corresponding upper 6; 3 remain. | ||
| + | |style="text-align:right;"|נחסיר ג' התחתונה מו' העליונה שכנגדה וישאר ג‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Erase the 6 and write the remaining 3 instead; erase also the 3 that is left beneath. | ||
| + | |style="text-align:right;"|נמחוק הו' ונכתוב במקומה הג' הנשארת ונמחק כמו כן הג' [שנשאר]‫<ref>M om.</ref> שלמטה | ||
| + | |- | ||
| + | | | ||
| + | ::It is like this: | ||
| + | |style="text-align:right;"|ויהיה כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |3||5||4 | ||
| + | |- | ||
| + | | ||2||1 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ג||ה||ד | ||
| + | |- | ||
| + | | ||ב||א | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Again, we subtract the bottom 2 from the corresponding upper 5; 3 remain. | ||
| + | |style="text-align:right;"|שוב נחסיר ב' תחתונה ‫<ref>111r</ref>מה' עליונה שכנגדה וישאר ג‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Write the 3 instead of the 5 and erase the bottom 3 as well as the upper 5. | ||
| + | |style="text-align:right;"|אות' ג' נכתוב במקום הה' ונמחק הב' שלמט' [וגם ה' שלמעלה]‫<ref>M om.</ref> | ||
| + | |- | ||
| + | | | ||
| + | ::It is like this: | ||
| + | |style="text-align:right;"|ויהיה כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |3||3||4 | ||
| + | |- | ||
| + | | || ||1 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ג||ג||ד | ||
| + | |- | ||
| + | | || ||א | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Again, we subtract the bottom 1 from the corresponding upper 4; 3 remain. | ||
| + | |style="text-align:right;"|שוב נחסיר א' תחתונה מד' עליונה [שכנגדה]‫<ref>M om.</ref> וישאר ג'ג'ג‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Erase the upper 4 as well as the bottom 1 and write 3 instead of the upper 4. | ||
| + | |style="text-align:right;"|‫[ומחוק ד' שלמעלה וגם א' שלמטה וכתוב ג' במקום ד' שלמעלה]‫<ref>M om.</ref> | ||
| + | |- | ||
| + | | | ||
| + | ::It is like this: | ||
| + | |style="text-align:right;"|‫[ויהיה כזה]‫<ref>M om.</ref> | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |3||3||3 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ג||ג||ג | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::We find that when we subtract 321 from 654, only 333 remains. | ||
| + | |style="text-align:right;"|נמצא כשחסרנו אב"ג מדה"ו לא נשאר כ"א ג'ג'ג‫' | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | ::{| | ||
| + | |- | ||
| + | |<span style="color:purple">6</span>54||rowspan="2"|<math>\scriptstyle\xrightarrow{{\color{Purple}{6-3}}={\color{blue}{3}}}</math>||<span style="color:#0000FF>3</span><span style="color:purple">5</span>4||rowspan="2"|<math>\scriptstyle\xrightarrow{{\color{Purple}{5-2}}={\color{blue}{3}}}</math>||3<span style="color:#0000FF>3</span><span style="color:purple">4</span>||rowspan="2"|<math>\scriptstyle\xrightarrow{{\color{Purple}{4-1}}={\color{blue}{3}}}</math>||33<span style="color:#0000FF>3</span> | ||
| + | |- | ||
| + | |<span style="color:purple">3</span>21|| <span style="color:purple">2</span>1||  <span style="color:purple">1</span>|| | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | *If the digit of the subtrahend is larger than the digit of the subtracted in the corresponding rank | ||
| + | |style="text-align:right;"|‫[וכאשר תמצא שהאות העליון אשר בקשנו לחסר ממנו פחות מן האות התחתון שתרצ' לחסר | ||
| + | |- | ||
| + | | | ||
| + | :*{{#annot:956-867|155|GybI}}As if you wish to subtract 867 from 956, like this: | ||
| + | ::<math>\scriptstyle956-867</math> | ||
| + | |style="text-align:right;"|כגון אם תרצ' לחסר זוח מן והט כזה{{#annotend:GybI}} | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |9||5||6 | ||
| + | |- | ||
| + | |8||6||7 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ט||ה||ו | ||
| + | |- | ||
| + | |ח||ו||ז | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::How do you subtract larger from smaller, like this: 7 from 6, you cannot and likewise 6 from 5. | ||
| + | |style="text-align:right;"|היאך תחסור מרובה ממועט כזה ז' מן ו' לא תוכל וכן ו' מן ה‫' | ||
| + | |- | ||
| + | | | ||
| + | ::This is how you do it: take 1 from 5, which is next to 6, erase the 5 and write 4. | ||
| + | |style="text-align:right;"|בדרך זה תעשה טול א' מן ה' אשר אצל ו' ומחוק הה' וכתוב ד‫' | ||
| + | |- | ||
| + | | | ||
| + | ::How much does this 1 add up to with the 6? 16, because it is in the rank that is second to 6; so it is 16. Subtract the 7 on the bottom row from 16; 9 remains. | ||
| + | |style="text-align:right;"|אותו א' כמה עולה לחבירו עם הו' י"ו כי היא במעלה שנייה מן הו' הרי י"ו חסר ז' אשר בטור השפל מן י"ו וישאר ט‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Then, you need to subtract 6 from 4. Do the same as I instructed you: erase 1 from 9; it becomes 14 in the preceding rank. Subtract 6 from it; 8 remains. | ||
| + | |style="text-align:right;"|אחר כך צריך לחסר ו' מן ד' עשה ג"כ כמו שצויתיך מחוק א' מן ט' ויהיה במעלה שלפניו י"ד וחסר ממנו ו' וישאר ח' ול"ד זה שייך לשער הכפל קטון‫]‫<ref>M om.</ref> | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | ::{| | ||
| + | |- | ||
| + | |95<span style="color:purple">6</span>||rowspan="2"|<math>\scriptstyle\xrightarrow{\begin{cases}\scriptstyle{\color{Purple}{16-7}}={\color{blue}{9}}\\\scriptstyle{\color{Purple}{5-1}}={\color{green}{4}}\end{cases}}</math>||[9<span style="color:#00FF00>4</span><span style="color:#0000FF>9</span>]||rowspan="2"|<math>\scriptstyle\xrightarrow{\begin{cases}\scriptstyle{\color{Purple}{14-6}}={\color{blue}{8}}\\\scriptstyle{\color{Purple}{9-1}}={\color{green}{8}}\end{cases}}</math>||[<span style="color:#00FF00>8</span><span style="color:#0000FF>8</span>9]||rowspan="2"|<math>\scriptstyle\xrightarrow{{\color{Purple}{8-8}}={\color{blue}{0}}}</math>||[89] | ||
| + | |- | ||
| + | |86<span style="color:purple">7</span>||[8<span style="color:purple">6</span> ]||[<span style="color:purple">8</span>  ]|| | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::I will teach you how to do it, because you cannot subtract 7 from 6 and also 6 from 5. This is how you do it: first, subtract the bottom 8 from the upper 9; 1 remains. Erase the 9 and write 1 in its place. Erase also the bottom 8. It is like this: | ||
| + | |style="text-align:right;"|‫<ref>MS Oxford 60, 153r-v</ref>אשכילך היאך תעשה כי לא תוכל לחסר ז' מן ו' וכן ו' מה' בדרך זה תעשה בתחילה חסר ח' תחתונה מט' עליונ' וישאר א' מחוק הט' וכתוב א' במקומ' גם ח' תחתונ' מחוק ויהיה כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |1||5||6 | ||
| + | |- | ||
| + | | ||6||7 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |א||ה||ו | ||
| + | |- | ||
| + | | ||ו||ז | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Now we need to subtract the bottom 6 from the upper 5 but we cannot. Therefore, go to the 1, which is to the left of the upper 5, and add together 1 and 5; it is 15. Subtract the 6 from it; 9 remains. Erase the upper 1 and the upper 5 also and write 9 in their place. Erase the bottom 6 as well. It remains like this: | ||
| + | |style="text-align:right;"|עתה צריכין אנו לחסר ו' תחתונ' מה' עליונ' ולא יכולנ' לכן לך לך אל הא' אשר לשמאל ה' עליונ' וצרפ' יחד א' וה' ויהיה ט"ו ונחסור הו' ממנו וישאר ט' מחוק הא' העליונ' גם ה' העליונ' וכתוב במקומם ט' גם ו' תחתונ' תמחוק וישאר כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |9||6 | ||
| + | |- | ||
| + | | ||7 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ט||ו | ||
| + | |- | ||
| + | | ||ז | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Again, we need to subtract the bottom 7 from 6, but we cannot. Now, go and take one from the 9 next to the 6 in the upper row and add together the 6 and the 1, which is ten to the 6; it is 16. Now subtract 7 from 16; 9 remains. Erase the upper 6 and 9 and write 9 and 8 in their place, as I instructed you. Erase the bottom 7 as well. It is like this: | ||
| + | |style="text-align:right;"|שוב צריכין אנו לחסר ז' תחתונ' מו' ולא יכולנ' עתה לך לך וקח אחד מן הט' שבצד הו' בשיט' עליונ' וצרפ' יחד הו' גם הא' והיא עשרה אצל הו' ויהיה י"ו ועתה חסר ז' מן י"ו וישאר ט' מחק ו' ט' עליונ' וכתוב ט' ח' במקומם כאשר ציויתיך ומחוק ג"כ הז' התחתונ' ויהיה כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |8||9 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ח||ט | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | ::{| | ||
| + | |- | ||
| + | |<span style="color:purple">9</span>56||rowspan="2"|<math>\scriptstyle\xrightarrow{{\color{Purple}{9-8}}={\color{blue}{1}}}</math>||<span style="color:#0000FF>1</span><span style="color:purple">5</span>6||rowspan="2"|<math>\scriptstyle\xrightarrow{\begin{cases}\scriptstyle{\color{Purple}{15-6}}={\color{blue}{9}}\\\scriptstyle{\color{Purple}{1-1=0}}\end{cases}}</math>||<span style="color:#0000FF>9</span><span style="color:purple">6</span>||rowspan="2"|<math>\scriptstyle\xrightarrow{\begin{cases}\scriptstyle{\color{Purple}{16-7}}={\color{blue}{9}}\\\scriptstyle{\color{Purple}{9-1}}={\color{blue}{8}}\end{cases}}</math>||<span style="color:#0000FF>89</span> | ||
| + | |- | ||
| + | |<span style="color:purple">8</span>67|| <span style="color:purple">6</span>7|| <span style="color:purple">7</span>|| | ||
| + | |} | ||
| + | |- | ||
| + | |The procedure is complete, look carefully. | ||
| + | |style="text-align:right;"|ותשלם המלאכה עיין היטב‫<ref>MS Oxford 60, 153r-v: end of the supplement</ref> | ||
| + | |- | ||
| + | | | ||
| + | === <span style=color:green>Checking Methods</span> === | ||
| + | |||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | *<span style=color:green>Addition</span> | ||
| + | :*If you want to know if you calculated correctly or not, add 321 to 333 according to the addition chapter: if you find 654 the subtraction is correct. | ||
| + | ::<math>\scriptstyle333+321=654</math> | ||
| + | |style="text-align:right;"|ואם תרצה לידע אם אמת חשבת אם לאו אז תחבר שער החיבור א'ב'ג' עם ג'ג'ג' ואם תמצא ד'ה'ו' אז החיסור מכוון | ||
| + | |- | ||
| + | | | ||
| + | :*If you want to know if you calculated correctly, add 867 to the remaining 89: if you find 956 you are right. | ||
| + | ::<math>\scriptstyle867+89=956</math> | ||
| + | |style="text-align:right;"|‫[ואם תרצ' לידע אם אמת חשבת אז חבר בשער החיבור ז'ו'ח' עם ט'ח' הנשארי' ואם תמצא ו'ה'ט' אז כיוונת]‫<ref>MS Oxford 60, 153v</ref> | ||
| + | |- | ||
| + | |This is the rule: the subtraction chapter is the scales for the addition chapter and the addition chapter is the scales for the subtraction chapter. This is enough for the one who understands. | ||
| + | |style="text-align:right;"|זה הכלל שער החיסור מאזני צדק לשער החיבור ושער החיבור מאזני צדק לשער החיסור ולמבין די | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | == Chapter Four: Multiplication == | ||
| + | |||
| + | |style="text-align:right;"|<big>שער הכפל גדול</big> | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:1234×4321|156|ZdlX}}If you wish to multiply 1234 by 4321. | ||
| + | :<math>\scriptstyle1234\times4321</math> | ||
| + | |style="text-align:right;"|אם בקשנו לכפול רל"ד ואלף על ד' אלפי' ושכ"א{{#annotend:ZdlX}} | ||
| + | |- | ||
| + | | | ||
| + | ::Here is its diagram, when you write one number next to the other, the first digit of the lower number corresponds to the last digit of the upper number, like this: | ||
| + | |style="width:45%; text-align:right;"|הילך צורתו כאשר תכתבנו חשבון האחד בצד השני ומכוון אות ראשון בחשבון תחתון תחת אות אחרון שבחשבון העליון כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | | || || ||4||3||2||1 | ||
| + | |- | ||
| + | |1||2||3||4|| || || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | | || || ||ד||ג||ב||א | ||
| + | |- | ||
| + | |א||ב||ג||ד|| || || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::We multiply the lower 1 by the upper 4, meaning multiply 1 times 4; it is only 4. | ||
| + | ::<math>\scriptstyle{\color{blue}{1\times4=4}}</math> | ||
| + | |style="text-align:right;"|נכפול א' תחתונה על ד' עליונה פי' נחשוב א' פעמי' ד' זה רק ד‫' | ||
| + | |- | ||
| + | | | ||
| + | ::We write 4 corresponding to the lower 1 and erase the lower 1, like this: | ||
| + | |style="text-align:right;"|אות' ד' נכתוב נגד א' תחתונה ונמחוק א' תחתונה כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |4|| || ||4||3||2||1 | ||
| + | |- | ||
| + | | ||2||3||4|| || || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ד|| || ||ד||ג||ב||א | ||
| + | |- | ||
| + | | ||ב||ג||ד|| || || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::We multiply also the lower 2 by the upper 4; the result is 8. | ||
| + | ::<math>\scriptstyle{\color{blue}{2\times4=8}}</math> | ||
| + | |style="text-align:right;"|עוד נכפול ב' תחתונה על ד' עליונה ויעלה ח‫' | ||
| + | |- | ||
| + | | | ||
| + | ::We write 8 corresponding to the lower 2 and erase the lower 2, like this: | ||
| + | |style="text-align:right;"|אות' ח' נכתוב נגד ב' התחתונה ונמחק ב' התחתונה כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |4||8|| ||4||3||2||1 | ||
| + | |- | ||
| + | | || ||3||4|| || || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ד||ח|| ||ד||ג||ב||א | ||
| + | |- | ||
| + | | || ||ג||ד|| || || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::We multiply also the lower 3 by the upper 4; the result is 12. | ||
| + | ::<math>\scriptstyle{\color{blue}{3\times4=12}}</math> | ||
| + | |style="text-align:right;"|עוד נכפול ג' תחתונה (על ד') על ד' עליונה ויעלה ב"א פי' י"ב | ||
| + | |- | ||
| + | | | ||
| + | ::Now, we write 2 corresponding to the lower 3 and 1 with the 8 that follows it, because it is ten, so it is one rank higher than the 2 before it; the result is 9. | ||
| + | ::<math>\scriptstyle{\color{blue}{1+8=9}}</math> | ||
| + | |style="text-align:right;"|עתה נכתוב הב' נגד ג' תחתונה והא' על הח' אחריה ויעלה ט' על כי הוא מעלה אחת שהיא עשרה אחר הב' שלפניה | ||
| + | |- | ||
| + | | | ||
| + | ::Erase the lower 3, like this: | ||
| + | |style="text-align:right;"|ומחוק ג' התחתונה כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |4||9||2||4||3||2||1 | ||
| + | |- | ||
| + | | || || ||4|| || || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ד||ט||ב||ד||ג||ב||א | ||
| + | |- | ||
| + | | || || ||ד|| || || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::We multiply also the lower 4 by the upper 4; the result is 16. | ||
| + | ::<math>\scriptstyle{\color{blue}{4\times4=16}}</math> | ||
| + | |style="text-align:right;"|עוד נכפול ד' תחתונה על ד' עליונה ויעלה ו"א [פי' י"ו]‫<ref>M om.</ref> | ||
| + | |- | ||
| + | | | ||
| + | ::Now, we write 6 corresponding to the lower 4, instead of the upper 4, since we have multiplied the upper 4 by the whole [multiplicand]. | ||
| + | |style="text-align:right;"|עתה נכתוב הו' נגד ד' התחתונה ובמקום ד' עליונה כי עתה נחשבנו הד' העליונ' בכל חלקיה | ||
| + | |- | ||
| + | | | ||
| + | ::We write the 1 with the 2 that follows it, so it becomes 3, like this: | ||
| + | ::<math>\scriptstyle{\color{blue}{1+2=3}}</math> | ||
| + | |style="text-align:right;"|ונכתוב הא' על הב' שאחריה ויהיה ג' כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |4||9||3||6||3||2||1 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ד||ט||ג||ו||ג||ב||א | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | ::<span style=color:green>[Illustration of the procedure:]</span> | ||
| + | ::{| | ||
| + | |- | ||
| + | |   <span style="color:purple">4</span>321||rowspan="2"|<math>\scriptstyle\xrightarrow{{\color{Purple}{4\times1}}={\color{blue}{4}}}</math>||<span style="color:#0000FF>4</span>  <span style="color:purple">4</span>321||rowspan="2"|<math>\scriptstyle\xrightarrow{{\color{Purple}{4\times2}}={\color{blue}{8}}}</math>||4<span style="color:#0000FF>8</span> <span style="color:purple">4</span>321||rowspan="2"|<math>\scriptstyle\xrightarrow{\begin{cases}\scriptstyle{\color{Purple}{4\times3}}={\color{green}{1}}{\color{blue}{2}}\\\scriptstyle{\color{Purple}{8+}}{\color{green}{1}}={\color{blue}{9}}\end{cases}}</math>||4<span style="color:#0000FF>92</span><span style="color:purple">4</span>321 | ||
| + | |- | ||
| + | |<span style="color:purple">1</span>234   || <span style="color:purple">2</span>34   ||  <span style="color:purple">3</span>4   ||   <span style="color:purple">4</span>    | ||
| + | |} | ||
| + | ::::{| | ||
| + | |- | ||
| + | |<math>\scriptstyle\xrightarrow{\begin{cases}\scriptstyle{\color{Purple}{4\times4}}={\color{green}{1}}{\color{blue}{6}}\\\scriptstyle{\color{Purple}{2+}}{\color{green}{1}}={\color{blue}{3}}\end{cases}}</math>||49<span style="color:#0000FF>36</span>321 | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::We shift 1234 one rank backwards, beneath the upper 3, in order to multiply the upper 3 by the whole [multiplicand] 1234, like this: | ||
| + | |style="text-align:right;"|ומעתה נמשך ד"גב"א מעלה לאחורית תחת ג' העליונה כדי לחשוב הג' [עליונה]‫<ref>M om.</ref> גם בכל תוצאותיה דגב"א כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |4||9||3||6||3||2||1 | ||
| + | |- | ||
| + | | ||1||2||3||4|| || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ד||ט||ג||ו||ג||ב||א | ||
| + | |- | ||
| + | | ||א||ב||ג||ד|| || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::We multiply the lower 1 by the upper 3, meaning 3 times 1; it is only 3. | ||
| + | ::<math>\scriptstyle{\color{blue}{1\times3=3}}</math> | ||
| + | |style="text-align:right;"|נכפול א' תחתונה על ג' עליונה פי' ג' פעמי' א' זהו רק ג‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Add 3 to the 9 above the lower 1; the result is 12. | ||
| + | ::<math>\scriptstyle{\color{blue}{3+9=12}}</math> | ||
| + | |style="text-align:right;"|ושים אות' ג' על ט' שעל הא' התחתונה ויעלה ב"א פי' י"ב | ||
| + | |- | ||
| + | | | ||
| + | ::We write 2 instead of the 9. | ||
| + | |style="text-align:right;"|עת' נכתוב ב' במקום ט‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Add the 1, which is ten, to the second rank, meaning the 4; the 4 becomes 5. | ||
| + | ::<math>\scriptstyle{\color{blue}{1+4=5}}</math> | ||
| + | |style="text-align:right;"|וא' שהיא עשרה שים אותה במעלה שנייה דהיינו בתוך הד' ונעשה הד' האחרונה ה‫' | ||
| + | |- | ||
| + | | | ||
| + | ::We erase the lower 1, like this: | ||
| + | |style="text-align:right;"|ונמחק הא' התחתונה כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |5||2||3||6||3||2||1 | ||
| + | |- | ||
| + | | || ||2||3||4|| || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ה||ב||ג||ו||ג||ב||א | ||
| + | |- | ||
| + | | || ||ב||ג||ד|| || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::We multiply also the lower 2 by the upper 3, 2 times 3; it is 6. | ||
| + | ::<math>\scriptstyle{\color{blue}{2\times3=6}}</math> | ||
| + | |style="text-align:right;"|עוד נכפול ב' תחתונה על ג' עליונה ב"פ"ג היינו ו‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Add the 6 to the 3 above it; it is 9. | ||
| + | ::<math>\scriptstyle{\color{blue}{6+3=9}}</math> | ||
| + | |style="text-align:right;"|ושים אות' ו' על ג' שעליה ויהיה ט‫' | ||
| + | |- | ||
| + | | | ||
| + | ::We erase the lower 2, like this: | ||
| + | |style="text-align:right;"|ונמחוק ב' תחתונה כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |5||2||9||6||3||2||1 | ||
| + | |- | ||
| + | | || || ||3||4|| || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ה||ב||ט||ו||ג||ב||א | ||
| + | |- | ||
| + | | || || ||ג||ד|| || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::We multiply also the lower 3 by the upper 3, 3 times 3; it is 9. | ||
| + | ::<math>\scriptstyle{\color{blue}{3\times3=9}}</math> | ||
| + | |style="text-align:right;"|עוד נכפול ג' תחתונה על ג' עליונה ג'פ"ג ט‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Add 9 to the 6 above it; the result is 15. | ||
| + | ::<math>\scriptstyle{\color{blue}{9+6=15}}</math> | ||
| + | |style="text-align:right;"|ושים אות' ט' על הו' שעליה ויעלה ה'א‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Write 5 instead of the 6 and the 1 in the second rank with the 9 that follows; it becomes 10. | ||
| + | ::<math>\scriptstyle{\color{blue}{1+9=10}}</math> | ||
| + | |style="text-align:right;"|הה' תכתוב במקום הו' והא' במעלה שנייה דהיינו אל הט' שאחריה ויהיה י‫' | ||
| + | |- | ||
| + | | | ||
| + | ::However, we should not write 10, because 10 is not written in the numeral calculation, only up to 9. So, we write a wheel, like this 0 and we write 1 in the following rank, meaning with the 2, so it becomes 3. | ||
| + | ::<math>\scriptstyle{\color{blue}{1+2=3}}</math> | ||
| + | |style="text-align:right;"|אך אין ראוי לכתוב י' כי אין ‫<ref>111v</ref>כותבין בחשבונות הציפרא י' רק עד ט' לכן נכתוב גלגל כזה 0 במקצוה ונכתוב א' במעלה של אחריה דהיינו אל הב' ועתה יהיה ג‫' | ||
| + | |- | ||
| + | | | ||
| + | ::We erase the lower 3, like this: | ||
| + | |style="text-align:right;"|ונמחוק ג' תחתונה כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |5||3||0||5||3||2||1 | ||
| + | |- | ||
| + | | || || || ||4|| || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ה||ג||0||ה||ג||ב||א | ||
| + | |- | ||
| + | | || || || ||ד|| || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::We multiply also the lower 4 by the upper 3; the result is 12. | ||
| + | ::<math>\scriptstyle{\color{blue}{4\times3=12}}</math> | ||
| + | |style="text-align:right;"|עוד נכפול <s>ג</s><sup>ד'</sup> תחתונה על ג' עליונה ויעלה ב"א | ||
| + | |- | ||
| + | | | ||
| + | ::Now, we write 2 instead of the upper 3, since we multiplied the 3 by the whole [multiplicand] and we write 1 in the second rank, with the 5; it becomes 6. | ||
| + | ::<math>\scriptstyle{\color{blue}{1+5=6}}</math> | ||
| + | |style="text-align:right;"|עתה נכתוב הב' במקום הג' העליונה כי נחשבנו ג"כ הג' בכל חלקיה והא' נכתוב במעלה שנייה אל הה' ויהיה ו‫' | ||
| + | |- | ||
| + | | | ||
| + | ::We erase the lower 4, like this. | ||
| + | |style="text-align:right;"|ונמחק ד' תחתונה כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |5||3||0||6||2||2||1 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ה||ג||0||ו||ב||ב||א | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | ::<span style=color:green>[Illustration of the procedure:]</span> | ||
| + | ::{| | ||
| + | |- | ||
| + | |4936<span style="color:purple">3</span>21||rowspan="2"|<math>\scriptstyle\xrightarrow{\begin{cases}\scriptstyle{\color{Purple}{3\times1}}={\color{green}{3}}\\\scriptstyle{\color{Purple}{9+}}{\color{green}{3}}={\color{green}{1}}{\color{blue}{2}}\\\scriptstyle{\color{Purple}{4+}}{\color{green}{1}}={\color{blue}{5}}\end{cases}}</math>||<span style="color:#0000FF>52</span>36<span style="color:purple">3</span>21||rowspan="2"|<math>\scriptstyle\xrightarrow{\begin{cases}\scriptstyle{\color{Purple}{3\times2}}={\color{green}{6}}\\\scriptstyle{\color{Purple}{3+}}{\color{green}{6}}={\color{blue}{9}}\end{cases}}</math>||52<span style="color:#0000FF>9</span>6<span style="color:purple">3</span>21||rowspan="2"|<math>\scriptstyle\xrightarrow{\begin{cases}\scriptstyle{\color{Purple}{3\times3}}={\color{green}{9}}\\\scriptstyle{\color{Purple}{6+}}{\color{green}{9}}={\color{green}{1}}{\color{blue}{5}}\\\scriptstyle{\color{Purple}{9+}}{\color{green}{1}}={\color{green}{1}}{\color{blue}{0}}\\\scriptstyle{\color{Purple}{2+}}{\color{green}{1}}={\color{blue}{3}}\end{cases}}</math>||5<span style="color:#0000FF>305</span><span style="color:purple">3</span>21 | ||
| + | |- | ||
| + | | <span style="color:purple">1</span>234  ||  <span style="color:purple">2</span>34  ||   <span style="color:purple">3</span>4  ||    <span style="color:purple">4</span>   | ||
| + | |} | ||
| + | ::::{| | ||
| + | |- | ||
| + | |<math>\scriptstyle\xrightarrow{\begin{cases}\scriptstyle{\color{Purple}{3\times4}}={\color{green}{1}}{\color{blue}{2}}\\\scriptstyle{\color{Purple}{5+}}{\color{green}{1}}={\color{blue}{6}}\end{cases}}</math>||530<span style="color:#0000FF>62</span>21 | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Now, we shift 1234 one rank backwards, beneath the upper 2, like this: | ||
| + | |style="text-align:right;"|ועתה נמשיך דגב"א מעלה אחת לאחוריו תחת הב' העליונה כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |5||3||0||6||2||2||1 | ||
| + | |- | ||
| + | | || ||1||2||3||4|| | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ה||ג||0||ו||ב||ב||א | ||
| + | |- | ||
| + | | || ||א||ב||ג||ד|| | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::We multiply the lower 1 by the upper 2, 1 times 2; it is 2. | ||
| + | ::<math>\scriptstyle{\color{blue}{1\times2=2}}</math> | ||
| + | |style="text-align:right;"|נכפול א' תחתונה על ב' עליונה א"פ"ב היינו ב‫' | ||
| + | |- | ||
| + | | | ||
| + | ::We write 2 corresponding to the lower 1, instead of the zero. | ||
| + | |style="text-align:right;"|אותה ב' נכתוב נגד א' תחתונה במקום הגלגל | ||
| + | |- | ||
| + | | | ||
| + | ::We erase the lower 1, like this: | ||
| + | |style="text-align:right;"|ונמחק א' תחתונה כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |5||3||2||6||2||2||1 | ||
| + | |- | ||
| + | | || || ||2||3||4|| | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ה||ג||ב||ו||ב||ב||א | ||
| + | |- | ||
| + | | || || ||ב||ג||ד|| | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::We multiply again the lower 2 by the upper 2, 2 times 2; it is 4. | ||
| + | ::<math>\scriptstyle{\color{blue}{2\times2=4}}</math> | ||
| + | |style="text-align:right;"|שוב נכפול ב' תחתונה על ב' עליונה ב"פ"ב' היינו ד‫' | ||
| + | |- | ||
| + | | | ||
| + | ::We write 4 above, corresponding to the lower 2, meaning with the 6; it is 10. | ||
| + | ::<math>\scriptstyle{\color{blue}{4+6=10}}</math> | ||
| + | |style="text-align:right;"|אות' ד' נכתוב למעלה נגד ב' תחתונה דהיינו אל הו' הרי י‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Write a zero instead of the 6 and add 1 after the zero, meaning to the 2; it is like this: | ||
| + | ::<math>\scriptstyle{\color{OliveGreen}{1+2=3}}</math> | ||
| + | |style="text-align:right;"|כתוב במקום הו' גלגל ואחר הגלגל [תוסי]ף א' דהיינו אל הב' ויהיה כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |5||3||3||0||2||2||1 | ||
| + | |- | ||
| + | | || || || ||3||4|| | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ה||ג||ג||0||ב||ב||א | ||
| + | |- | ||
| + | | || || || ||ג||ד|| | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::We multiply again the lower 3 by the upper 2, 2 times 3; it is 6. | ||
| + | ::<math>\scriptstyle{\color{blue}{3\times2=6}}</math> | ||
| + | |style="text-align:right;"|שוב נכפול ג' תחתונה על ב' עליונה ב"פ"ג היינו ו‫' | ||
| + | |- | ||
| + | | | ||
| + | ::We write 6 corresponding to the lower 3, meaning with the upper 2; it becomes 8. | ||
| + | ::<math>\scriptstyle{\color{blue}{6+2=8}}</math> | ||
| + | |style="text-align:right;"|אות' ו' נכתוב נגד ג' תחתונה דהיינו אל הב' [עליונה]‫<ref>M om.</ref> ויהיה ח‫' | ||
| + | |- | ||
| + | | | ||
| + | ::We erase the lower 3, like this: | ||
| + | |style="text-align:right;"|ונמחק הג' התחתונה ויהיה כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |5||3||3||0||8||2||1 | ||
| + | |- | ||
| + | | || || || || ||4|| | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ה||ג||ג||0||ח||ב||א | ||
| + | |- | ||
| + | | || || || || ||ד|| | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::We multiply again the lower 4 by the upper 2, 2 times 4; it is 8. | ||
| + | ::<math>\scriptstyle{\color{blue}{4\times2=8}}</math> | ||
| + | |style="text-align:right;"|שוב נכפול ד' תחתונה על הב' העליונה ב"פ"ד ח‫' | ||
| + | |- | ||
| + | | | ||
| + | ::We write 8 instead of the upper 2, since we have multiplied the 2 by the whole [multiplicand] and we erase the lower 4, like this: | ||
| + | |style="text-align:right;"|נכתוב ח' במקום ב' עליונה כי גם [חשבנו]‫<ref>M: חשבון</ref> הב' בכל חלקיה ונמחק ד' תחתונה כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |5||3||3||0||8||8||1 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ה||ג||ג||0||ח||ח||א | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | ::<span style=color:green>[Illustration of the procedure:]</span> | ||
| + | ::{| | ||
| + | |- | ||
| + | |53062<span style="color:purple">2</span>1||rowspan="2"|<math>\scriptstyle\xrightarrow{{\color{Purple}{2\times1=}}{\color{blue}{2}}}</math>||53<span style="color:#0000FF>2</span>62<span style="color:purple">2</span>1||rowspan="2"|<math>\scriptstyle\xrightarrow{\begin{cases}\scriptstyle{\color{Purple}{2\times2}}={\color{green}{4}}\\\scriptstyle{\color{Purple}{6+}}{\color{green}{4}}={\color{green}{1}}{\color{blue}{0}}\\\scriptstyle{\color{Purple}{2+}}{\color{green}{1}}={\color{blue}{3}}\end{cases}}</math>||53<span style="color:#0000FF>30</span>2<span style="color:purple">2</span>1||rowspan="2"|<math>\scriptstyle\xrightarrow{\begin{cases}\scriptstyle{\color{Purple}{2\times3}}={\color{green}{6}}\\\scriptstyle{\color{Purple}{2+}}{\color{green}{6}}={\color{blue}{8}}\end{cases}}</math>||5330<span style="color:#0000FF>8</span><span style="color:purple">2</span>1 | ||
| + | |- | ||
| + | |  <span style="color:purple">1</span>234 ||   <span style="color:purple">2</span>34 ||    <span style="color:purple">3</span>4 ||     <span style="color:purple">4</span>  | ||
| + | |} | ||
| + | ::::{| | ||
| + | |- | ||
| + | |<math>\scriptstyle\xrightarrow{\scriptstyle{\color{Purple}{2\times4}}={\color{blue}{8}}}</math>||53308<span style="color:#0000FF>8</span>1 | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::We shift 1234 another rank backwards, in order to multiply it by the first 1, like this: | ||
| + | |style="text-align:right;"|נמשך דגב"א עוד מעלה אחת לאחוריו כדי לכפול אות' <s>ב</s> אותה על א' הראשונה ויהיה כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |5||3||3||0||8||8||1 | ||
| + | |- | ||
| + | | || || ||1||2||3||4 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ה||ג||ג||0||ח||ח||א | ||
| + | |- | ||
| + | | || || ||א||ב||ג||ד | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::We multiply the lower 1 by the upper 1, 1 times 1; it is 1. | ||
| + | ::<math>\scriptstyle{\color{blue}{1\times1=1}}</math> | ||
| + | |style="text-align:right;"|נכפול א' תחתונה על א' עליונה‫<ref>M: הראשונה</ref> א"פ"א היינו א‫' | ||
| + | |- | ||
| + | | | ||
| + | ::We write 1 corresponding to the lower 1, instead of the zero, and erase the lower 1, like this: | ||
| + | |style="text-align:right;"|אותה א' נכתוב נגד א' התחתונה במקום הגלגל ונמחק א' תחתונה כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |5||3||3||1||8||8||1 | ||
| + | |- | ||
| + | | || || || ||2||3||4 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ה||ג||ג||א||ח||ח||א | ||
| + | |- | ||
| + | | || || || ||ב||ג||ד | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::We multiply again the lower 2 by the upper 1, 2 times 1; it is 2. | ||
| + | ::<math>\scriptstyle{\color{blue}{2\times1=2}}</math> | ||
| + | |style="text-align:right;"|שוב נכפול ב' תחתונה על א' עליונה ב"פ"א היינו ב‫' | ||
| + | |- | ||
| + | | | ||
| + | ::We write 2 above the lower 2, meaning with the 8: we add 2 to 8; it is 10. | ||
| + | ::<math>\scriptstyle{\color{blue}{2+8=10}}</math> | ||
| + | |style="text-align:right;"|אות' ב' נכתו' על הב' התחתונה דהיינו אל הח' נוסיף ב' על הח' ויהיה י‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Write a zero instead of the 8. | ||
| + | |style="text-align:right;"|כתוב במקום הח' גלגל ואחר הגלגל | ||
| + | |- | ||
| + | | | ||
| + | ::We add 1 to the 1; it is 2. | ||
| + | ::<math>\scriptstyle{\color{blue}{1+1=2}}</math> | ||
| + | |style="text-align:right;"|נוסיף א' על הא' ויהיה ב‫' | ||
| + | |- | ||
| + | | | ||
| + | ::We erase the lower 2, like this: | ||
| + | |style="text-align:right;"|ונמחק ב' התחתונה כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |5||3||3||2||0||8||1 | ||
| + | |- | ||
| + | | || || || || ||3||4 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ה||ג||ג||ב||0||ח||א | ||
| + | |- | ||
| + | | || || || || ||ג||ד | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::We multiply again the lower 3 by the upper 1, 3 times 1; it is 3. | ||
| + | ::<math>\scriptstyle{\color{blue}{3\times1=3}}</math> | ||
| + | |style="text-align:right;"|שוב נכפול ג' תחתונה על א' עליונה ג"פ"א היינו ג‫' | ||
| + | |- | ||
| + | | | ||
| + | ::We write 3 corresponding to the lower 3, meaning with 8; it is 11. | ||
| + | ::<math>\scriptstyle{\color{blue}{3+8=11}}</math> | ||
| + | |style="text-align:right;"|אות' ג' נכתוב נגד ג' תחתונה דהיינו ח' הרי י"א | ||
| + | |- | ||
| + | | | ||
| + | ::We erase the 8. We write 1 instead of it and 1 instead of the zero. | ||
| + | |style="text-align:right;"|נמחוק הח' ונכתוב במקומה א' ואחריה במקום הגלגל א‫' | ||
| + | |- | ||
| + | | | ||
| + | ::We erase the lower 3, like this: | ||
| + | |style="text-align:right;"|ונמחק ג' תחתונה כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |5||3||3||2||1||1||1 | ||
| + | |- | ||
| + | | || || || || || ||4 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ה||ג||ג||ב||א||א||א | ||
| + | |- | ||
| + | | || || || || || ||ד | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::We multiply also the lower 4 by the upper 1, 4 times 1; it is 4. | ||
| + | ::<math>\scriptstyle{\color{blue}{4\times1=4}}</math> | ||
| + | |style="text-align:right;"|שוב נכפול ד' תחתונה אל א' עליונה ד"פ"א היינו ד‫' | ||
| + | |- | ||
| + | | | ||
| + | ::We write 4 instead of the corresponding 1, but we do not add 1 to the 4, as I have instructed you before, because we just multiplied 1234 by this 1. This 1 only indicated the number of times, not for adding. | ||
| + | |style="text-align:right;"|אות' ד' נכתוב במקום א' שכנגדה אבל לא נצטרף אות' א' עם הד' כמו שצויתיך כבר כי על אות' א' כפלנו עכשיו [דגב"א ול]א היתה הא' רק לסימן כמה פעמי' לכן לא חדייה לצירוף | ||
| + | |- | ||
| + | | | ||
| + | ::We erase the lower 4, like this: | ||
| + | |style="text-align:right;"|ונמחק ד' תחתונה כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |5||3||3||2||1||1||4 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ה||ג||ג||ב||א||א||ד | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::We find that 4321 times 1234 is 5332114, meaning 5 hundred and 33 tens of thousands, 2 thousandths, one hundred and 14. | ||
| + | |style="text-align:right;"|נמצא כי אבג"ד פעמי' דגב"א עולה בחשבון ד'א'א'ב'ג'ג'ה' דהיינו י"ד ומאה אחת וב' אלפים ול"ג ריבואות וה' מאות ריבואות וד"ל | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | ::<span style=color:green>[Illustration of the procedure:]</span> | ||
| + | ::{| | ||
| + | |- | ||
| + | |533088<span style="color:purple">1</span>||rowspan="2"|<math>\scriptstyle\xrightarrow{\begin{cases}\scriptstyle{\color{Purple}{1\times1}}={\color{green}{1}}\\\scriptstyle{\color{Purple}{0+}}{\color{green}{1}}={\color{blue}{1}}\end{cases}}</math>||533<span style="color:#0000FF>1</span>88<span style="color:purple">1</span>||rowspan="2"|<math>\scriptstyle\xrightarrow{\begin{cases}\scriptstyle{\color{Purple}{1\times2}}={\color{green}{2}}\\\scriptstyle{\color{Purple}{8+}}{\color{green}{2}}={\color{green}{1}}{\color{blue}{0}}\\\scriptstyle{\color{Purple}{1+}}{\color{green}{1}}={\color{blue}{2}}\end{cases}}</math>||533<span style="color:#0000FF>20</span>8<span style="color:purple">1</span>||rowspan="2"|<math>\scriptstyle\xrightarrow{\begin{cases}\scriptstyle{\color{Purple}{1\times3}}={\color{green}{3}}\\\scriptstyle{\color{Purple}{8+}}{\color{green}{3}}={\color{blue}{11}}\end{cases}}</math>||5332<span style="color:#0000FF>11</span><span style="color:purple">1</span> | ||
| + | |- | ||
| + | |   <span style="color:purple">1</span>234||    <span style="color:purple">2</span>34||     <span style="color:purple">3</span>4||      <span style="color:purple">4</span> | ||
| + | |} | ||
| + | ::::{| | ||
| + | |- | ||
| + | |<math>\scriptstyle\xrightarrow{\scriptstyle{\color{Purple}{1\times4}}={\color{blue}{4}}}</math>||533211<span style="color:#0000FF>4</span> | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:209×3030|156|Lq1V}}Another example: we wish to multiply 209 by 3030. | ||
| + | :<math>\scriptstyle209\times3030</math> | ||
| + | |style="text-align:right;"|‫<ref>112r</ref>דומיון אחר הנה בקשנו לכפול ט' ומאתים על ג' אלפי' ול‫'{{#annotend:Lq1V}} | ||
| + | |- | ||
| + | | | ||
| + | ::This is how you write it diagram: | ||
| + | |style="text-align:right;"|הילך היאך תכתוב צורתו כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | | || ||3||0||3||0 | ||
| + | |- | ||
| + | |2||0||9|| || || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | | || ||ג||0||ג||0 | ||
| + | |- | ||
| + | |ב||0||ט|| || || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::We multiply the lower 2 by the upper 3, 2 times 3; it is 6. | ||
| + | ::<math>\scriptstyle{\color{blue}{2\times3=6}}</math> | ||
| + | |style="text-align:right;"|נכפול ב' התחתונה על ג' עליונה ב"פ"ג היינו ו‫' | ||
| + | |- | ||
| + | | | ||
| + | ::We write 6 corresponding to the lower 2 and erase the lower 2. | ||
| + | |style="text-align:right;"|אות' ו' נכתוב ב' נגד ב' תחתונה ונמחק ב' תחתונה | ||
| + | |- | ||
| + | | | ||
| + | ::Erase also the zero before the lower 2 and write a zero before the 6, because we multiply a zero times [zero]; it is a zero, like this: | ||
| + | ::<math>\scriptstyle{\color{blue}{0\times0=0}}</math> | ||
| + | |style="text-align:right;"|וגם הגלגל שלפני הב' התחתונ' מחוק וכתוב גלגל לפני הו' כזה כי נכפול גלגל פעמי' ויהיה גלגל | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |6||0||3||0||3||0 | ||
| + | |- | ||
| + | | || ||9|| || || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ו||0||ג||0||ג||0 | ||
| + | |- | ||
| + | | || ||ט|| || || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::We multiply again 9 by 3 above it, 9 times 3; it is 27. | ||
| + | ::<math>\scriptstyle{\color{blue}{9\times3=27}}</math> | ||
| + | |style="text-align:right;"|שוב נכפול ט' על ג' שעליה ט"פ"ג היינו כ"ז | ||
| + | |- | ||
| + | | | ||
| + | ::We write 27 above instead of the last 3; it is 7 instead of the 3 and 2 instead of the zero. | ||
| + | |style="text-align:right;"|ואות' כ"ז נכתוב למעלה במקום הג' האחרונה ויהיה ז' במקום הג' וב' במקום הגלגל | ||
| + | |- | ||
| + | | | ||
| + | ::We erase the 9, like this: | ||
| + | |style="text-align:right;"|ונמחק ט' התחתונה כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |6||2||7||0||3||0 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ו||ב||ז||0||ג||0 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | ::<span style=color:green>[Illustration of the procedure:]</span> | ||
| + | ::{| | ||
| + | |- | ||
| + | |  <span style="color:purple">3</span>030||rowspan="2"|<math>\scriptstyle\xrightarrow{{\color{Purple}{3\times2}}={\color{blue}{6}}}</math>||<span style="color:#0000FF>6</span>0<span style="color:purple">3</span>030||rowspan="2"|<math>\scriptstyle\xrightarrow{{\color{Purple}{3\times9}}={\color{blue}{27}}}</math>||6<span style="color:#0000FF>27</span>0<span style="color:purple">3</span>0 | ||
| + | |- | ||
| + | |<span style="color:purple">2</span>09   ||  <span style="color:purple">9</span>   || | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::We shift 209 two ranks backwards, so that the lower 9, which is the first digit in the lower line will correspond the upper 3, like this: | ||
| + | |style="text-align:right;"|נחזור ט0"ב לאחוריו ב' מעלות כדי שתהא ט' התחתונה שהוא אות ראשונה שבשיטה תחתונה נגד ג' עליונה כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |6||2||7||0||3||0 | ||
| + | |- | ||
| + | | || ||2||0||9|| | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ו||ב||ז||0||ג||0 | ||
| + | |- | ||
| + | | || ||ב||0||ט|| | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::If you want to know why we do not write 209 beneath the upper zero, of course we do so, but in any case it does not yield more than a zero, because if there are digits written and you need to add a zero to them it does not add to them, as will be explained below. | ||
| + | |style="text-align:right;"|וא"ת למה לא כתבם ט'0'ב' תחת הגלגל העליון ודאי עשינו כך אבל מ"מ לא עולה יותר מגלגל כי אם תמצא אותיות כתובות ואתה צריך לצרף עמהם גלגל אין צורפי' עמהם ול"ד כמו שפי' לקמן | ||
| + | |- | ||
| + | | | ||
| + | ::We multiply the lower 2 by the upper 3, 2 times 3; it is 6. | ||
| + | ::<math>\scriptstyle{\color{blue}{2\times3=6}}</math> | ||
| + | |style="text-align:right;"|נכפול ב' התחתונה על ג' עליונה ב"פ"ג היינו ו‫' | ||
| + | |- | ||
| + | | | ||
| + | ::We write 6 corresponding to the lower 2, meaning with the 7; it is 13. and erase the lower 2. | ||
| + | ::<math>\scriptstyle{\color{blue}{6+7=13}}</math> | ||
| + | |style="text-align:right;"|אות' ו' נכתוב נגד ב' תחתונה דהיינו אל הז' ויהיה י"ג | ||
| + | |- | ||
| + | | | ||
| + | ::We erase the 7 and write 3 instead of it. | ||
| + | |style="text-align:right;"|נמחק הז' ונכתוב במקומה ג‫' | ||
| + | |- | ||
| + | | | ||
| + | ::We add 1 to the 2 that follows it, because it is ten, as I wrote above. | ||
| + | ::<math>\scriptstyle{\color{OliveGreen}{1+2=3}}</math> | ||
| + | |style="text-align:right;"|ואחריה נוסיף א' על ב' כי היא עשרה כמו שכתבתי לעיל | ||
| + | |- | ||
| + | | | ||
| + | ::We erase 2 and we erase also the zero before the 2. | ||
| + | |style="text-align:right;"|ונמחק ב' וגם הגלגל שלפני הב' נמחק | ||
| + | |- | ||
| + | | | ||
| + | ::We do not need to write a zero above, as you already did, since there is already a zero corresponding to it. All the more so if there were a non-zero digit [in the upper result line] corresponding the zero, we would not need not erase this digit in order to write a zero, because, if we erase a non-zero digit, in order to write a zero there, we decrease [the numerical value of the rank], and there is no need [for the zero] to hold the place, since the a non-zero digit is already holding the place. | ||
| + | |style="text-align:right;"|ואין אנו צריכי' לצייר אותו גלגל למעלה כמו שעשית כבר כי בלאו הכי יש גלגל כנגדו וכ"ש אם היה אות נגד הגלגל שלא היינו מוחק' האות לצייר שום גלגל שאם היינו מוחק' האות כדי לצייר שם גלגל אז היינו ממעטין החשבון ואם לעשות כדי להרחיק המעלה זה אינו כי בלאו הכי האות מרחיק המעלה | ||
| + | |- | ||
| + | | | ||
| + | ::In short: we never write the lower zero above, unless there is nothing that corresponds it. | ||
| + | |style="text-align:right;"|כללא דמילתא לעולם אין אנו מציירין גלגל התחתון למעלה אלא אם אין כנגדו שום דבר | ||
| + | |- | ||
| + | | | ||
| + | ::But, if there is a zero or a non-zero digit corresponding to the lower zero, we do not need to write a zero [instead], since it is drawn only as a place holder, as I wrote above, because the zero indicates that digit that follows it is of one rank higher than it would have been, had you not written a zero there. | ||
| + | |style="text-align:right;"|אבל אם יש שום גלגל [או אות]‫<ref>M om.</ref> נגד אותו גלגל התחתון אז אין אנו צריכין לצייר שום גלגל כי ציורו אינו אלא [{{#annot:|233,2451|pxwf}}שומר המעלה{{#annotend:pxwf}}]‫<ref>M: מעלה העלמא</ref> כמו שכתבתי לעיל [כי הגלגל מודיע שאות אחריו עולה מעלה אחת יותר ממה שהיית עושה אם לא היית שם שום ציור גלגל‫]‫<ref>M om.</ref> | ||
| + | |- | ||
| + | | | ||
| + | ::Returning to the above calculation, when you do as I explained, it is like this: | ||
| + | |style="text-align:right;"|ועתה נחזור לחשבון דלעיל כשתעשה כמו שפירשתי אז יהיה כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |6||3||3||0||3||0 | ||
| + | |- | ||
| + | | || || || ||9|| | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ו||ג||ג||0||ג||0 | ||
| + | |- | ||
| + | | || || || ||ט|| | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::We multiply again the lower 9 by the upper 3, 9 times 3; it is 27. | ||
| + | ::<math>\scriptstyle{\color{blue}{9\times3=27}}</math> | ||
| + | |style="text-align:right;"|שוב נכפול ט' תחתונה על ג' עליונה ט"פ"ג היינו כ"ז | ||
| + | |- | ||
| + | | | ||
| + | ::We write 7 instead of the upper 3, then 2 instead of the zero. We erase the lower 9 and also the upper 3, like this: | ||
| + | |style="text-align:right;"|נכתוב במקום ג' עליונה ז' ואח"כ במקום גלגל ב' ונמחק ט' תחתון וגם הג' העליונה [מח]ק כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |6||3||3||2||7||0 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ו||ג||ג||ב||ז||0 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::We find that two hundred and 9 times 3 thousand and 30 is 63 tens of thousands, 3 thousand, two hundred and seventy. | ||
| + | |style="text-align:right;"|נמצא שבמאתים וט' פעמי' ג' אלפי' ול' יש ס"ג רבבות וג' אלפי' ומאתים ושבעי‫' | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | ::<span style=color:green>[Illustration of the procedure:]</span> | ||
| + | ::{| | ||
| + | |- | ||
| + | |6270<span style="color:purple">3</span>0||rowspan="2"|<math>\scriptstyle\xrightarrow{\begin{cases}\scriptstyle{\color{Purple}{3\times2}}={\color{green}{6}}\\\scriptstyle{\color{Purple}{7+}}{\color{green}{6}}={\color{green}{1}}{\color{blue}{3}}\\\scriptstyle{\color{Purple}{2+}}{\color{green}{1}}={\color{blue}{3}}\end{cases}}</math>||6<span style="color:#0000FF>33</span>0<span style="color:purple">3</span>0||rowspan="2"|<math>\scriptstyle\xrightarrow{{\color{Purple}{3\times9}}={\color{blue}{27}}}</math>||633<span style="color:#0000FF>27</span>0 | ||
| + | |- | ||
| + | |  <span style="color:purple">2</span>09 ||    <span style="color:purple">9</span> || | ||
| + | |} | ||
| + | |- | ||
| + | |This belongs to the chapter on multiplication. | ||
| + | |style="text-align:right;"|‫<ref>125v</ref>וזהו שייך לשער הכפל | ||
| + | |- | ||
| + | |I will show you another way to multiply: | ||
| + | |style="text-align:right;"|<big>הנה</big> אראך בדרך אחר לכפול | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:15×1080|156|5pXt}}If you wish to know how much are 15 times 1080. | ||
| + | :<math>\scriptstyle15\times1080</math> | ||
| + | |style="text-align:right;"|אם תרצה [לידע]‫<ref>M om.</ref> כמה ט"ו פעמי' תתר"ף{{#annotend:5pXt}} | ||
| + | |- | ||
| + | | | ||
| + | ::Write 1080 in the first line and 15 beneath it - 5 beneath the 1 and 1 to its left, like this: | ||
| + | |style="text-align:right;"|עשה טור ראשון מתתר"ף ושים תחתיו ט"ו ה' תחת הא' וא' לשמאלו כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | | ||1||0||8||0 | ||
| + | |- | ||
| + | |1||5|| || || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | | ||א||0||ח||0 | ||
| + | |- | ||
| + | |א||ה|| || || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Next, multiply the 1 by the entire upper line, then the 5 by the entire upper line: | ||
| + | |style="text-align:right;"|אז תכפול הא' על כל הטור העליון ואח"כ ה' על כל הטור העליון | ||
| + | |- | ||
| + | | | ||
| + | ::Say: 1 times 1 is 1. | ||
| + | ::<math>\scriptstyle{\color{blue}{1\times1=1}}</math> | ||
| + | |style="text-align:right;"|וכה תאמר אפ"א הרי א‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Write 1 corresponding to the bottom 1 of the multiplier, so that it will be above the 1080, corresponding to the bottom 1. The diagram is like this: | ||
| + | |style="text-align:right;"|שים א' כנגד א' השפל מן הכפלות כל כך גבוה שיהא הוא למעלה מן התתר"ף [מכוון כנגד הא' התחתונ' ויהיה צורת' כזה]‫<ref>M om.</ref> | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |1|| || || || | ||
| + | |- | ||
| + | | ||1||0||8||0 | ||
| + | |- | ||
| + | |1||5|| || || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |א|| || || || | ||
| + | |- | ||
| + | | ||א||0||ח||0 | ||
| + | |- | ||
| + | |א||ה|| || || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Then, multiply 1 times zero; it is zero. | ||
| + | ::<math>\scriptstyle{\color{blue}{1\times0=0}}</math> | ||
| + | |style="text-align:right;"|ואח"כ כפול א' פעמי' גלגל הרי גלגל | ||
| + | |- | ||
| + | | | ||
| + | ::Write a zero before the 1 you wrote above the 1080, which corresponds to the bottom 1 in the first row. Like this: | ||
| + | |style="text-align:right;"|שים גלגל לפני הא' אשר כתבתי למעלה מן התתר"ף [מכוונ' נגד הא' בשיט' ראשונ' ויהיה כזה]‫<ref>M om.</ref> | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |1||0|| || || | ||
| + | |- | ||
| + | | ||1||0||8||0 | ||
| + | |- | ||
| + | |1||5|| || || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |א||0|| || || | ||
| + | |- | ||
| + | | ||א||0||ח||0 | ||
| + | |- | ||
| + | |א||ה|| || || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Next, multiply 1 times 8; the result is 8. | ||
| + | ::<math>\scriptstyle{\color{blue}{1\times8=8}}</math> | ||
| + | |style="text-align:right;"|ואח"כ כפול א' פעמי' ח' יעלה ח‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Write 8 before the zero you wrote in the upper row, corresponding to the zero in the middle row. Like this: | ||
| + | |style="text-align:right;"|כתוב ח' לפני ה0' שכתבת [בשיט' עליונ' מכוונת נגד הציפר' שבשיט' אמצעי' ויהיה כזה]‫<ref>M om.</ref> | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |1||0||8|| || | ||
| + | |- | ||
| + | | ||1||0||8||0 | ||
| + | |- | ||
| + | |1||5|| || || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |א||0||ח|| || | ||
| + | |- | ||
| + | | ||א||0||ח||0 | ||
| + | |- | ||
| + | |א||ה|| || || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Then, multiply 1 times 0; the result is 0. | ||
| + | ::<math>\scriptstyle{\color{blue}{1\times0=0}}</math> | ||
| + | |style="text-align:right;"|ואח"כ כפול אפ"0 ויעלה 0‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Write it before the 8 you wrote in the upper row, which corresponds to the zero in the middle row. | ||
| + | |style="text-align:right;"|שימהו לפני הח' אשר כתבת [בשיט' עליונ' מכוון נגד 0' שבשיט' אמצעי‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Erase the 1, as it has been multiplied by the whole 1080. It is like this: | ||
| + | |style="text-align:right;"|גם תמחוק הא' כי נכפל על כל התתר"ף]‫<ref>M om.</ref> ויהיה כזה דמיונו | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |1||0||8||0|| | ||
| + | |- | ||
| + | | ||1||0||8||0 | ||
| + | |- | ||
| + | | ||5|| || || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |א||0||ח||0|| | ||
| + | |- | ||
| + | | ||א||0||ח||0 | ||
| + | |- | ||
| + | | ||ה|| || || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::You have multiplied the 1 by the whole 1080. | ||
| + | |style="text-align:right;"|והנה כפלת הא' על כל התתר"ף | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | ::<span style=color:green>[Illustration of the procedure:]</span> | ||
| + | ::{| | ||
| + | |- | ||
| + | | ||rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{Purple}{1\times1}}={\color{blue}{1}}}</math>||<span style="color:#0000FF>1</span>    ||rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{Purple}{1\times0}}={\color{blue}{0}}}</math>||1<span style="color:#0000FF>0</span>   ||rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{Purple}{1\times8}}={\color{blue}{8}}}</math>||10<span style="color:#0000FF>8</span>  ||rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{Purple}{1\times0}}={\color{blue}{0}}}</math>||108<span style="color:#0000FF>0</span>  | ||
| + | |- | ||
| + | | <span style="color:purple">1</span>080|| 1<span style="color:purple">0</span>80|| 10<span style="color:purple">8</span>0|| 108<span style="color:purple">0</span>|| 1080 | ||
| + | |- | ||
| + | |<span style="color:purple">1</span>5   ||<span style="color:purple">1</span>5   ||<span style="color:purple">1</span>5   ||<span style="color:purple">1</span>5   ||15    | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Next, multiply the 5 by the whole 1080. | ||
| + | |style="text-align:right;"|ואח"כ כפול הה' על כל התתרף | ||
| + | |- | ||
| + | | | ||
| + | ::Start multiplying it by it right above: as you started multiplying the 1 by 1, so multiply the 5. | ||
| + | |style="text-align:right;"|ותתחיל לכופלו כנגדו ממש למעלה כאשר התחלת לכפול הא' כנגד [א']‫<ref>M om.</ref> כן תכפול הה‫' | ||
| + | |- | ||
| + | | | ||
| + | ::5 times 1 is 5. | ||
| + | ::<math>\scriptstyle{\color{blue}{5\times1=5}}</math> | ||
| + | |style="text-align:right;"|ה' פ"א הוי ה‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Write 5 corresponding to the 1, above it, that is instead of the zero, like this: | ||
| + | |style="text-align:right;"|שים ה' נגד הא' למעלה הימנו דהיינו במקום גלגל [כזה]‫<ref>M om.</ref> | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |1||5||8||0|| | ||
| + | |- | ||
| + | | ||1||0||8||0 | ||
| + | |- | ||
| + | | ||5|| || || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |א||ה||ח||0|| | ||
| + | |- | ||
| + | | ||א||0||ח||0 | ||
| + | |- | ||
| + | | ||ה|| || || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Then, say: 5 times 0 [is 0]. | ||
| + | ::<math>\scriptstyle{\color{blue}{5\times0=0}}</math> | ||
| + | |style="text-align:right;"|ואח"כ אמור הפ"0 | ||
| + | |- | ||
| + | | | ||
| + | ::If nothing was written above the zero, you would write a zero there. Now that 8 is written above it, you do not do anything with the zero, because it holds its place. | ||
| + | |style="text-align:right;"|אם לא היה נכתב מאומה על הגלגל היית נותן שם גלגל עכשיו שרשום עליו ח' לא תעשה מהגלגל כלום כי אוחז מקומו | ||
| + | |- | ||
| + | | | ||
| + | ::Then, say: 5 times 8; the result is forty. | ||
| + | ::<math>\scriptstyle{\color{blue}{5\times8=40}}</math> | ||
| + | |style="text-align:right;"|ואח"כ אמור הפ"ח יעלה ארבעים | ||
| + | |- | ||
| + | | | ||
| + | ::Do not write it instead of the zero, because you need the zero, but write 4 above the 8 to the left, then you will find 8 and 4 on top of each other. Erase both of them and write 2 in their place; on the 5 that you wrote to the left write 1; it is 6, like this: | ||
| + | |style="text-align:right;"|ולא תשימהו במקום הגלגל כי תצטרך לגלגל אלא כתוב ד' על הח' לשמאל אז תמצא ד"ח זה על זה תמחוק שניהם ותכתוב במקומו ב' ועל הה' אשר כתבת לשמאל כתוב א' הרי ו' [כזה]‫<ref>M om.</ref> | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |1||6||2||0|| | ||
| + | |- | ||
| + | | ||1||0||8||0 | ||
| + | |- | ||
| + | | ||5|| || || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |א||ו||ב||0|| | ||
| + | |- | ||
| + | | ||א||0||ח||0 | ||
| + | |- | ||
| + | | ||ה|| || || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Then, say: 5 times zero is a zero. | ||
| + | ::<math>\scriptstyle{\color{blue}{5\times0=0}}</math> | ||
| + | |style="text-align:right;"|ואח"כ אמור ה"פ גלגל היינו גלגל | ||
| + | |- | ||
| + | | | ||
| + | ::Write a zero above the zero. It is like this: | ||
| + | |style="text-align:right;"|שים גלגל על גלגל ויהא דמיונו כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |1||6||2||0||0 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |א||ו||ב||0||0 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | ::<span style=color:green>[Illustration of the procedure:]</span> | ||
| + | ::{| | ||
| + | |- | ||
| + | |1080 ||rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{Purple}{5\times1}}={\color{blue}{5}}}</math>||1<span style="color:#0000FF>5</span>80 ||rowspan="3"|<math>\scriptstyle\xrightarrow{\begin{cases}\scriptstyle{\color{Purple}{5\times0}}={\color{green}{0}}\\\scriptstyle{\color{Purple}{8+}}{\color{green}{0}}={\color{blue}{8}}\\\end{cases}}</math>||15<span style="color:#0000FF>8</span>0 ||rowspan="3"|<math>\scriptstyle\xrightarrow{\begin{cases}\scriptstyle{\color{Purple}{5\times8}}={\color{green}{4}}{\color{blue}{0}}\\\scriptstyle{\color{Purple}{8+}}{\color{green}{4}}={\color{green}{1}}{\color{blue}{2}}\\\scriptstyle{\color{Purple}{5+}}{\color{green}{1}}={\color{blue}{6}}\\\end{cases}}</math>||1<span style="color:#0000FF>620</span> ||rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{Purple}{5\times0}}={\color{blue}{0}}}</math>||1620<span style="color:#0000FF>0</span> | ||
| + | |- | ||
| + | | <span style="color:purple">1</span>080|| 1<span style="color:purple">0</span>80|| 10<span style="color:purple">8</span>0|| 108<span style="color:purple">0</span>|| | ||
| + | |- | ||
| + | |1<span style="color:purple">5</span>   ||1<span style="color:purple">5</span>   ||1<span style="color:purple">5</span>   ||1<span style="color:purple">5</span>   || | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | :Understand that the upper row that corresponds to the bottom row is not complete until you multiply all the digits of the multiplier by the whole number. | ||
| + | |style="text-align:right;"|תבין שלא נשלם הטור העליון נגד הטור השפל עד אשר תכפול כל האותיות מן הכפולות על כל החשבון ול"י סליק | ||
| + | |- | ||
| + | | | ||
| + | === <span style=color:green>Checking Methods</span> === | ||
| + | | | ||
| + | |- | ||
| + | |If you are in doubt saying who said this is so, here are scales to check whether you were wrong or not: | ||
| + | |style="text-align:right;"|‫<ref>112r continue</ref>ואם לבך מפקפק לומר מאן יימר שכך [הוא]‫<ref>M om.</ref> הילך מאזנים היאך {{#annot:term|2141,2449|YQDx}}תשקול{{#annotend:YQDx}} ותבחין אם טעית אם לאו | ||
| + | |- | ||
| + | | | ||
| + | *<span style=color:green>Casting out 9 by 9</span> | ||
| + | :First take the number you wanted to multiply in the upper row, cast out the nines and keep the remainder. | ||
| + | |style="text-align:right;"|בראשונה תקח חשבון שבשיטה העליונה אשר <s>ב</s> בקשת לכפול והשלך ‫<ref>112v</ref>אותו ט'ט' ט'ט' והמותר קח בידך | ||
| + | |- | ||
| + | | | ||
| + | :If you do not find 9 even once, take it all. | ||
| + | |style="text-align:right;"|ואם לא תמצא אפילו פעם אחת [ט']‫<ref>M om.</ref> אז תקח הכל | ||
| + | |- | ||
| + | | | ||
| + | :Then, take the bottom number and cast out the nines also. | ||
| + | |style="text-align:right;"|ואח"כ תיקח החשבון התחתונה והשלך ג"כ אז בט"ט | ||
| + | |- | ||
| + | | | ||
| + | :Take what you have left and multiply it according to the multiplication chapter by what you have left from the upper row after you cast out the nines from it. Cast out the nines from the result you get as well and keep the remainder that is less than 9. | ||
| + | |style="text-align:right;"|ומה שישאר בידך קח נא והשלך אותו בשער הכפל על מה שנשאר לך בשיטה עליונה אחר שהשלכת ממנו ט'ט' ומה שיעלה בידך השלך אותו ג"כ בט"ט ומה שנשאר שלא הגיע לכלל ט' קח נא בידך | ||
| + | |- | ||
| + | | | ||
| + | :Then, take the [original] result you got when you multiplied the two numbers together and cast out the nines from its as well. | ||
| + | |style="text-align:right;"|ואח"כ קח החשבון היוצא לך כבר כשכפלת הב' חשבונות יחד והשלך אותו ג"כ בט"ט ט"ט | ||
| + | |- | ||
| + | | | ||
| + | :If what remains in your hand is the same as what you have left, know that you calculated correctly, otherwise you must have been mistaken. | ||
| + | |style="text-align:right;"|ומה שנשאר בידך אם הוא מכוון כנגד מה שנשאר לך כבר ידוע תדע שיפה חשבנת ואם לאו בודאי טעית | ||
| + | |- | ||
| + | | | ||
| + | :Now, we will check the above calculation, so that the one who understands will understand for sure and ''increase in learning'' [Proverbs 1, 5]: | ||
| + | |style="text-align:right;"|ועתה {{#annot:term|2141,2449|QPGO}}נשקול{{#annotend:QPGO}} חשבון דלעיל למען יבין המבין ויוסיף לקח | ||
| + | |- | ||
| + | | | ||
| + | :*The example: 3030 times 209 | ||
| + | ::<math>\scriptstyle209\times3030</math> | ||
| + | |style="text-align:right;"|הדמיון היה 0"ג0"ג פעמים ט0"ב | ||
| + | |- | ||
| + | | | ||
| + | ::First, we sum up 2 times 3, i.e. 6; there is no 9 even once. | ||
| + | ::<math>\scriptstyle{\color{blue}{3+0+3+0=3+3=6\equiv_96}}</math> | ||
| + | |style="text-align:right;"|בתחילה נחבר ב' פעמי' ג' היינו ו' ואין כאן אפילו פעם אחת ט‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Then, we sum up the bottom number, i.e. 209: we sum 9 and 2; it is 11. Subtract 9; 2 remains. | ||
| + | ::<math>\scriptstyle{\color{blue}{2+0+9=2+9=11\equiv_92}}</math> | ||
| + | |style="text-align:right;"|ואח"כ נחבר חשבון התחתון דהיינו ט0"ב נחבר ט'ב' היינו י"א<br> | ||
| + | השלך ט' וישאר ב‫' | ||
| + | |- | ||
| + | | | ||
| + | ::We multiply 2 by 6: 2 times 6 is 12. Subtract 9; 3 remains. This is the reserved. | ||
| + | ::<math>\scriptstyle{\color{blue}{6\times2=12\equiv_93}}</math> | ||
| + | |style="text-align:right;"|נכפול ב' על ו' <s>בפנ</s> בפ"ו היינו י"ב<br> | ||
| + | השלך ט' וישאר ג' וזה יהיה לך לזכרון | ||
| + | |- | ||
| + | | | ||
| + | ::This is the rule: see what is left for you from the product, as now when we were required to multiply 2 times 6, which is 12. Write 12 in digits; it is 12. The excess over 9 is 3. | ||
| + | ::<math>\scriptstyle{\color{blue}{6\times2=12\longrightarrow1+2=3}}</math> | ||
| + | |style="text-align:right;"|זה הכלל ראה בכפל מה נשאר לך כמו עתה הוצרכנו לכפול בפ"ו והיה י"ב <s>ו</s> כתוב י"ב בציפרא ויהיה ב"א היינו יתיר <s>מג</s> ואל יגיע על ט' ג' וכן כולם וד"ל | ||
| + | |- | ||
| + | | | ||
| + | ::Then, see the result you got when you multiplied 3030 by 209, i.e. 633270: we sum them up; it is 21. Cast out the nines; 3 remains, which is the same as what you already have left, so the calculation is correct. | ||
| + | |style="text-align:right;"|ואח"כ ראה מה שיעלה בידך כ<sup>ש</sup>כפלת 0'ג'0'ג' על ט'0'ב' דהיינו 0'ז'ב'ג'ג'ו‫'<br> | ||
| + | נחבר אותם יחד ויהיה כ"א<br> | ||
| + | השלך ט'ט' וישאר ג' כמו שנשאר לך כבר וא"כ החשבון מכוון ודו"ק | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | ::<math>\scriptstyle{\color{blue}{3030\times209=633270\longrightarrow6+3+3+2+7+0=21\equiv_93}}</math> | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | == Chapter [Five]: Multiplication in a Table [Gelosia] == | ||
| + | |||
| + | |style="text-align:right;"|<big>שער הכפל בסולם</big> | ||
| + | |- | ||
| + | |This diagram is called a table [lit. ladder] and it is appropriate for the multiplication chapter. | ||
| + | |style="text-align:right;"|<big>ציור</big> זה נקרא סולם ויפה לשער הכפל [גדול]‫<ref>M om.</ref> | ||
| + | |- | ||
| + | |As the size of the numbers you want to multiply by each other, draw the number of cells. | ||
| + | |style="text-align:right;"|וכמה שתרצה לכפול חשבון על חשבון כן עשה מניין הבתים | ||
| + | |- | ||
| + | | | ||
| + | :If you multiply a number of three ranks by a number of three ranks, draw three cells horizontally and vertically in the table. | ||
| + | |style="text-align:right;"|אם תכפול חשבון בג' מעלות על ג' מעלות כמו כן תצייר שליבות הסולם ג' בתים לארכה ולרחבה | ||
| + | |- | ||
| + | | | ||
| + | :If you multiply a number of three ranks by two ranks, draw three cells horizontally and two cells vertically. | ||
| + | |style="text-align:right;"|ואם תכפול סך בג' מעלות על ב' מעלות עשה ג' בתים לרחבה וב' בתים לארכה | ||
| + | |- | ||
| + | | | ||
| + | :And vice versa, from top to bottom, one larger and one smaller, provided that each digit is arranged in its place according to the diagram. | ||
| + | |style="text-align:right;"|וכן להפך מלמעלה למטה אחד המרבה ואחד הממעיט ובלבד שיכוון לבו כל אות' [במקומו]‫<ref>M on.</ref> לפי הציור | ||
| + | |- | ||
| + | | | ||
| + | :*{{#annot:321×654|156|lJrF}}Example: if you wish to know how much is 3 hundred and 21 times 654, written 321 by 654. | ||
| + | ::<math>\scriptstyle321\times654</math> | ||
| + | |style="text-align:right;"|דמיון אם תרצה לידע כמה כ"א וג' מאות פעמי' נ"ד ות"ר שרשימתו אב"ג דה"ו{{#annotend:lJrF}} | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | [[File:Ṣifra - Gelosia - 1.png|thumb|300px|center]] | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | [[File:ציפרא - כפל בסולם - א.png|thumb|300px|center]] | ||
| + | |- | ||
| + | | | ||
| + | ::Write above the cells, as you see in the table I wrote, and write the multiplicand on the side, one [digit] above the other, as illustrated. | ||
| + | |style="text-align:right;"|רשום למעלה על הבתים כאשר תראה בסולם שרשמתי והנכפול כתוב בצדו זה תחת זה כמצוייר | ||
| + | |- | ||
| + | | | ||
| + | ::<span style=color:green>First line</span> | ||
| + | :::First, multiply 4 time 1; the result is 4. Write it in the half of the first cell, beneath the 1, next to the 4. | ||
| + | :::<math>\scriptstyle{\color{blue}{4\times1=4}}</math> | ||
| + | |style="text-align:right;"|<s>וב</s> ובראשונה חשוב כמה ד' פעמי' א' ויעלה ד' וכתוב אותו בחצי בית ‫<ref>113r</ref>ראשון שמכוון תחת הא' <s>ונגד</s> נגד הד‫' | ||
| + | |- | ||
| + | | | ||
| + | :Because every cell is divided in half: its upper half is for the units [of the interim product] and the bottom [half] is for the tens. | ||
| + | |style="text-align:right;"|כי כל בית נחלק מחציתו העליון אחדים והתחתוני' עשירים | ||
| + | |- | ||
| + | | | ||
| + | :::Then, multiply 4 times 2; the result is 8. Write it in the half of the [second] upper cell, beneath the 2. | ||
| + | :::<math>\scriptstyle{\color{blue}{4\times2=8}}</math> | ||
| + | |style="text-align:right;"|ואח"כ חשוב ד' פעמי' ב' ויעלה ח' רשמיהו בחצי בית העליון שתחת ב‫' | ||
| + | |- | ||
| + | | | ||
| + | :::Next, multiply 4 times 3; the result is 12. Write it in the upper cell beneath the 3: write 2 in the upper half and 1 in the other half. | ||
| + | :::<math>\scriptstyle{\color{blue}{4\times3=12}}</math> | ||
| + | |style="text-align:right;"|ואח"כ חשוב כמה ד'פ'ג' ויעלה ב'א' רשמיהו באותו בית שתחת הג' וכתוב ב' <sup>בחצי</sup> <s>באותו</s> חלק העליון וא' בחציי' השנייה | ||
| + | |- | ||
| + | | | ||
| + | :::Now, erase the 4, because it has been multiplied by all the parts. | ||
| + | |style="text-align:right;"|ומעתה תמחוק הד' כי היא נחשבת בכל חלוקיה | ||
| + | |- | ||
| + | | | ||
| + | ::<span style=color:green>Second line</span> | ||
| + | :::Now, multiply 5 time 1; the result is 5. Write it in the cell next to the 5 beneath the 1. | ||
| + | :::<math>\scriptstyle{\color{blue}{5\times1=5}}</math> | ||
| + | |style="text-align:right;"|מעתה תצטרך לחשוב הפ"א ויעל' ה' ורושומיה בבית שבצד הה' מכוון תח' הא‫' | ||
| + | |- | ||
| + | | | ||
| + | :::Then, multiply 5 times 2; the result is 10. Write it in the half of the fifth cell. | ||
| + | :::<math>\scriptstyle{\color{blue}{5\times2=10}}</math> | ||
| + | |style="text-align:right;"|ואח"כ חשוב הפ"ב ויעלה 0"א רשומיהו בחציי <sup>ה</sup>תחתון בבית חמישין | ||
| + | |- | ||
| + | | | ||
| + | :::Next, multiply 5 times 3; the result is 15. Write it in the sixth cell: 5 in the upper half and 1 in the lower half. | ||
| + | :::<math>\scriptstyle{\color{blue}{5\times3=15}}</math> | ||
| + | |style="text-align:right;"|ואח"כ חשוב הפ"ג ויעלה ה"א רשומיה בבית שישי [ה' בחצי העליון א' בחצי תחתון]‫<ref>M om.</ref> | ||
| + | |- | ||
| + | | | ||
| + | :::Now, erase the 5. | ||
| + | |style="text-align:right;"|ומעתה תמחוק הה‫' | ||
| + | |- | ||
| + | | | ||
| + | ::<span style=color:green>Third line</span> | ||
| + | :::Multiply 6 times 1; the result is 6. Write it next to it in the column beneath the 1. | ||
| + | :::<math>\scriptstyle{\color{blue}{6\times1=6}}</math> | ||
| + | |style="text-align:right;"|והנה חשוב ופ"א יעלה ו' רשימהו בצידו מכוון בטור שתחת הא‫' | ||
| + | |- | ||
| + | | | ||
| + | :::6 times 2 is 12. Write it in the eighth cell. | ||
| + | :::<math>\scriptstyle{\color{blue}{6\times2=12}}</math> | ||
| + | |style="text-align:right;"|ו' פעמים ב' ב"א רשימיהו בבית <s>האחרון</s> <sup>השמיני</sup> | ||
| + | |- | ||
| + | | | ||
| + | :::6 times 3 is 18. Write it in the last cell. | ||
| + | :::<math>\scriptstyle{\color{blue}{6\times3=18}}</math> | ||
| + | |style="text-align:right;"|‫[ו' פ"ג היינו ח"א רשימיהו בבית האחרון]‫<ref>marg.</ref> | ||
| + | |- | ||
| + | | | ||
| + | ::We have multiplied all of it. | ||
| + | |style="text-align:right;"|והנה כפלנו כולו | ||
| + | |- | ||
| + | | | ||
| + | ::I will teach you how much is the total result: | ||
| + | |style="text-align:right;"|ואשכילך כמה יעלה סכום שלה | ||
| + | |- | ||
| + | | | ||
| + | ::Take the first digit in the first cell, which is 4, and write it in a special place. | ||
| + | |style="text-align:right;"|קח אות ראשון שבבית ראשון שהיא ד' ותכתביהו במקום מיוחד | ||
| + | |- | ||
| + | | | ||
| + | ::Then, calculate the digits on the second diagonal from 8 to 5: add 5 to 8; the result is 13. | ||
| + | ::<math>\scriptstyle{\color{blue}{5+8=13}}</math> | ||
| + | |style="text-align:right;"|ואח"כ חשוב בשליבה שנייה בכל השיפוע מאות ח' עד ה' וצרוף הה' אל הח' ויעלה ג"א | ||
| + | |- | ||
| + | | | ||
| + | ::Write the 3 next to the 4 that is already written, like this: | ||
| + | |style="text-align:right;"|הג' כתוב בצד הד' הנרשמת כבר כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |3||4 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ג||ד | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Add the 1, which is ten, to the next diagonal: add it to the 2 at the beginning of the third diagonal; the result is 3. Then, add also the 6 at the end of the diagonal; it is 9. | ||
| + | ::<math>\scriptstyle{\color{blue}{1+2+6=3+6=9}}</math> | ||
| + | |style="text-align:right;"|והא' שהיא עשרה צרוף לשליבה שתחתיו וחבריהו אל הב' בתחיל' שליבה שלישית ויעלה ג' וחבר אח"כ גם הו' שבסוף השיפוע ויהיה ט‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Write it next to the 4 and the 3, like this: | ||
| + | |style="text-align:right;"|ורשמיהו אצל ד"ג כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |9||3||4 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ט||ג||ד | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Then, sum up the digits on the fourth diagonal from the top to the end; the result is 9. Write is next to 9, 3, 4 like this: | ||
| + | |style="text-align:right;"|ואח"כ חשוב וצרוף אותיות שבשליבה רביעי' מראש' ועד סופ' ויעלה ט' וכתביהו בצד דג"ט כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |9||9||3||4 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ט||ט||ג||ד | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Sum up the digits on the fifth diagonal; the resulting sum is ten. | ||
| + | |style="text-align:right;"|והנה חשוב אותיות שבשליבה חמישית בשיפועו ויעלה צירופו עשרה | ||
| + | |- | ||
| + | | | ||
| + | :I already said that the tens are added to the diagonal below and are not written in the special place. | ||
| + | |style="text-align:right;"|וכבר אמרתי שלעולם העשירי' מצטרפ' לשליבה שתחתיו ואין רושמי' אותה בצד המיוחדי‫' | ||
| + | |- | ||
| + | | | ||
| + | ::But, you should write a zero in the place of the ten in order to hold the rank, since there is not even one left to the indicate the rank. | ||
| + | |style="text-align:right;"|אבל תצטרך להשי' גלגל במקום העשרה לשמור המעלו' הואיל ואין נשאר אפילו אחד [לשמור]‫<ref>M om.</ref> המורה המעלה | ||
| + | |- | ||
| + | | | ||
| + | ::When you add the ten to the 1 on the last diagonal, the result is 2. Write it next to the special 9, 9, 3, 4, like this: 209934. | ||
| + | |style="text-align:right;"|והנה כש{{#annot:term|178,1677|8C34}}תצטרף{{#annotend:8C34}} העשרה לא' שבשליב' אחרונה יעלה ב' וכתביהו בצד דגט"ט המיוחדי' כזה ד ג ט ט 0 ב | ||
| + | |- | ||
| + | | | ||
| + | ::This is the correct calculation of multiplying 321 by 654. | ||
| + | |style="text-align:right;"|זה מכוון חשבון של כפולתם אב"ג על דה"ו | ||
| + | |- | ||
| + | | | ||
| + | ::Its scales are as I wrote above in the multiplication chapter. | ||
| + | |style="text-align:right;"|ומאזנים שלו כמו שכתבתי לעיל בשער הכפל [הגדול]‫<ref>M om.</ref> | ||
| + | |- | ||
| + | | | ||
| + | :*{{#annot:464×464|156|bkz3}}464 times 464: the result is 215296. | ||
| + | ::<math>\scriptstyle464\times464</math> | ||
| + | |style="text-align:right;"|דו"ד פעמי' דו"ד יעלה חשבונו וט"בהא"ב ודו"ק{{#annotend:bkz3}} | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | [[File:Ṣifra - Gelosia - 2.png|thumb|300px|center]] | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | [[File:ציפרא - כפל בסולם - ב.png|thumb|300px|center]] | ||
| + | |- | ||
| + | |The multiplication chapter is complete. | ||
| + | |style="text-align:right;"|סליק שער הכפל | ||
| + | |- | ||
| + | | | ||
| + | === <span style=color:green>Multiplication - Check</span> === | ||
| + | | | ||
| + | |- | ||
| + | |When you want to know the scales, if the result is correct, when you have the product: | ||
| + | |style="text-align:right;"|‫<ref>MS Mantova f. 69r</ref>כשתרצה לידע משקל אם אתא שפיר כשיש לפניך הכפל | ||
| + | |- | ||
| + | | | ||
| + | *As 464 times 464 once, which is 215296, or twice: is it correct or not. | ||
| + | :<math>\scriptstyle464\times464=215296</math> | ||
| + | |style="text-align:right;"|כגו' א דוד פעם דוד כגו' ו'ט'ב'ה'א'ב' או כל כפלים אם הן שפיר או לאו | ||
| + | |- | ||
| + | | | ||
| + | :Then, take the sum of 4, 6, 4; we find it is 14. | ||
| + | |style="text-align:right;"|אז קח לפניך דוד במניין נמצא י"ד | ||
| + | |- | ||
| + | | | ||
| + | :Say: how much greater than 9 is it? We find it is 5. Cast out the 9. | ||
| + | |style="text-align:right;"|ואמ' כמה הוא יותר מט' נמצא ה' ותשליך הט' ממך | ||
| + | |- | ||
| + | | | ||
| + | :Say: how much is 5 times 5? We find it is 25. | ||
| + | |style="text-align:right;"|ואמ' כמה ה' פעמי' ה' נמצא כ"ה | ||
| + | |- | ||
| + | | | ||
| + | :Cast out all the nines that are in 25; you are left with 7. | ||
| + | |style="text-align:right;"|ותשליך ממך כל הט' שבתוך כ"ה ויתר לך ז‫' | ||
| + | |- | ||
| + | | | ||
| + | :<math>\scriptstyle{\color{blue}{\scriptstyle\begin{cases}\scriptstyle4+6+4=14\equiv_95\\\scriptstyle4+6+4=14\equiv_95\end{cases}\scriptstyle\longrightarrow5\times5=25\equiv_97}}</math> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :Then, cast out the nines found in 215296 as well; you are left with 7. | ||
| + | :<math>\scriptstyle{\color{blue}{215296\equiv_97}}</math> | ||
| + | |style="text-align:right;"|אז תשליך נמי ו'ט'ב'ה'א'ב' בט' אז ישאר לך ז‫' | ||
| + | |- | ||
| + | |Do the same with all the products in the world. | ||
| + | |style="text-align:right;"|וכן תעשה לכל הכפולים שבעולם ודו"ק | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | == Chapter Six: Division == | ||
| + | |||
| + | |style="width:45%; text-align:right;"|‫<ref>113r continue</ref><big>שער החילוק</big> | ||
| + | |- | ||
| + | |The chapter on division is appropriate for dividing a large number into a few smaller numbers | ||
| + | |style="text-align:right;"|<big>שער החילוק</big> והוא טוב לחלק חשבון גדול לכמה חשבונות קטנים | ||
| + | |- | ||
| + | |You should know that in this chapter the first digit of the bottom row is not written corresponding to the last digit of the top row, as in the multiplication chapter, but the top row is written according to its ranks and the bottom row [is also written] according to its ranks, so that the last digit of the bottom row corresponds to the last digit of the top row. | ||
| + | |style="text-align:right;"|ועתה ידוע תדע שאין כותבין בשער זה כמו בשער כפל אות ראשונה משורה תחתונה נגד אות אחרונה משורה עליונה אלא כותבין שורה עליונה כפי מעלותיה ושורה תחתונה כמו כן כפי מעלותיה ויכוין אות אחרונה שבשורה תחתונה נגד אות אחרונה שבשורה עליונה | ||
| + | |- | ||
| + | |If the last digit of the bottom row [= divisor] is greater than the last digit of the upper row [= dividend], the bottom row is shifted one rank backwards, unless the bottom row has more than one digit. | ||
| + | |style="text-align:right;"|‫[ואם אות אחרונה שבשורה תחתונה יותר מאות אחרונה שבשורה עליונה]‫<ref>M om.</ref> אז יסוג שורה תחתונה מעלה אחת לאחוריה [אלא א"כ דאותו שורה תחתונה יש בה יותר מאות אחת]‫<ref>M om.</ref> | ||
| + | |- | ||
| + | |If they [= the last digits of the two rows] are the same [the bottom row] should not be shifted, unless it has more than one digit. | ||
| + | |style="text-align:right;"|אבל אם עלו שוות לא יסוג לאחריה אלא ‫<ref>113v</ref>א"כ דאותו שורה יש בה יותר מאות אחת‫<ref>marg.:נאם מאיר גראשוואל ודוק המה ולפי עניות דעתי אינו אחור זה החשבון מכמה טעמי' ונימוקים אלא כך היא החשבון ובקלות נמרצת דהיינו אם תרצה לחשוב כמה פעמי' ז' יש במאתים וי"ח בחשבון תכתוב כזה ותכתוב הז' תחת הא' ותאמר כמה פעמי' ז' יש בעשרים ואחד דהיינו ג' פעמים וכתוב אות' ג' מן הצד כזה ותמחוק הא"ב והז' ואז כזה ואז יסוג הז' תחת הח' ותאמר כמה פעמים כמה פעמים ז' יש בח' תחתונה דהיינו א' פעמים וכתוב אות' א' מן הצד אצל ג' כזה וישארו<br> | ||
| + | נראה למהר"ר ווייזל היינו דווק' כשאות' אות שלפני האחרונה בשור' התחתונה יתיר' על אות' אות שלפני האחרונה בשור' העליונה כזה : הג"ה</ref> | ||
| + | |- | ||
| + | |When it has more that one digit, the whole row should be shifted one rank backwards, so that the upper digits can be divided by the lower digits. | ||
| + | |style="text-align:right;"|וכשיש בה יותר מאות אחת יסוג כל השורה מעלה אחת לאחוריה ואז יכול לחלק אותיות עליונות על אותיות תחתונות | ||
| + | |- | ||
| + | |The quotient is written corresponding to the first rank of the lower row, whether this rank is a non-zero digit, or a zero. | ||
| + | |style="text-align:right;"|והחילוק שחילקו יכתוב נגד מעלה ראשונה שבשורה תחתונה הן אם אות' מעלה אות או גלגל | ||
| + | |- | ||
| + | |When you divide by 2 digit, meaning that there are 2 digits in the bottom row, both are [subtracted] the same [number of times], whether [this number consists of] 3 digits, or 4 and so on endlessly, as the number of times you take the last digit of the number, so is the number of times you take the rest of the digits. | ||
| + | |style="text-align:right;"|וכשתחלוק ב' אותיו' פי' שהיו בשור' תחתונה ב' אותיות אז אין חילוק של שתיהן רק מניין אחד [יהיה]‫<ref>M: הה'</ref> ג' אותיו' או ד' וכן עד סוף כל הדורות כל כמה פעמים שלקחת האות האחרונה מן החשבון כך תקח כל שאר האותיות | ||
| + | |- | ||
| + | |This is enough to understand; examine and you will find [that it is true]. | ||
| + | |style="text-align:right;"|ול"ד ודו"ק ודו"ק ול"ד וד"ל ול"ד | ||
| + | |- | ||
| + | |Here is the chapter of division, suitable for everything and also for dividing a large number into several small numbers. | ||
| + | |style="text-align:right;"|<big>ו</big>הילך שער החילוק וטוב לכל דבר וכן עמ' דבר לחלק חשבון גדול לכמה חשבונות קטנים | ||
| + | |- | ||
| + | !<span style=color:green>Examples</span> | ||
| + | | | ||
| + | |- | ||
| + | |I shall write examples so that one will understand. | ||
| + | |style="text-align:right;"|ועתה אכתוב דמיונים למען יבין המבין | ||
| + | |- | ||
| + | |''Instruct a wise man, and he will become wiser... and increase in learning'' [Proverbs 9, 9]. | ||
| + | |style="text-align:right;"|{{#annot:Pr9-9|494|S63h}}''ותן לחכם ויחכם עוד'' ''ויוסיף לקח''{{#annotend:S63h}}‫<ref group=note>משלי ט, ט</ref> | ||
| + | |- | ||
| + | |Now I start dividing by a small number: | ||
| + | |style="text-align:right;"|ועתה אתחיל בחילוק קטן | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:218÷7|157|vVRf}}If one asks: how many times 7 is in two hundred and 18? | ||
| + | :<math>\scriptstyle218\div7</math> | ||
| + | |style="text-align:right;"|אם ישאל השואל כמה פעמי' ז' יש במאתים וי"ח{{#annotend:vVRf}} | ||
| + | |- | ||
| + | | | ||
| + | ::Here is how you do it: | ||
| + | |style="text-align:right;"|הילך היאך תעשה | ||
| + | |- | ||
| + | | | ||
| + | ::Write two hundred and 18 like this: | ||
| + | |style="text-align:right;"|תכתוב המאתים וי"ח ויהיה כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |2||1||8 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ב||א||ח | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::The 7 should have been written beneath the 2, in order to divide the 2 by 7, meaning to count how many times 7 is in 2, but 2 cannot be divided by 7. Therefore the 7 is shifted backwards, so that the 2 is in the second rank to it and it becomes twenty-one corresponding to it, like this: | ||
| + | |style="text-align:right;"|והז' היה מן הדין לכתוב תחת הב' כדי לחלק הב' לז' ז' פי' למנה כמה פעמים ז' יש בב' אלא שאין הב' מגיע לחילוק של ז' לכן נסוג הז' לאחור כדי שתהא הב' במעלה שנייה [לה]‫<ref>M om.</ref> ותעלה לנגדה עשרים ואחד כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |2||1||8 | ||
| + | |- | ||
| + | | ||7|| | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ב||א||ח | ||
| + | |- | ||
| + | | ||ז|| | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Divide twenty-one by 7, meaning count how many times 7 is in twenty-one. We find that there are 3 times 7 in it. | ||
| + | ::<math>\scriptstyle{\color{blue}{21\div7=3}}</math> | ||
| + | |style="text-align:right;"|ואותן עשרים ואחד תחלוק לז' ז' פי' מנה כמה פעמי' ז' יש בעשרי' ואחד ונמצא שיש בה ג' פעמי' ז‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Write 3 above the 1 in the upper row, corresponding the 7 in the lower row, like this: | ||
| + | |style="text-align:right;"|ואותה ג' כתוב למעלה על הא' שבשורה עליונה נגד ז' שבשורה התחתונה כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | | ||3|| | ||
| + | |- | ||
| + | |2||1||8 | ||
| + | |- | ||
| + | | ||7|| | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | | ||ג|| | ||
| + | |- | ||
| + | |ב||א||ח | ||
| + | |- | ||
| + | | ||ז|| | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Erase the 21, in which you find the 3 times 7, then the 7 is shifted one rank backwards, beneath the 8, like this: | ||
| + | |style="text-align:right;"|ואח"כ תמחוק הא"ב שמצאת בהם ג' פעמי' ז' ואח"כ יסוג הז' מעלה אחת לאחוריה ויהיה תחת הח' כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |3|| | ||
| + | |- | ||
| + | | ||8 | ||
| + | |- | ||
| + | | ||7 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ג|| | ||
| + | |- | ||
| + | | ||ח | ||
| + | |- | ||
| + | | ||ז | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Count how many times 7 is in 8; you find 7 only once and one remains. | ||
| + | ::<math>\scriptstyle{\color{blue}{8\div7=1+\frac{1}{8}}}</math> | ||
| + | |style="text-align:right;"|ומנה כמה פעמי' ז' יש בח' ולא נמצא בה רק פעם אחת ז' ואחד עודף | ||
| + | |- | ||
| + | | | ||
| + | ::So, write 1 corresponding to the 7, above the 8, and write the remaining 1 instead of the 8, like this: | ||
| + | |style="text-align:right;"|אז תכתוב א' נגד הז' על הח' כזה והאחד העודפת כתוב במקום הח' כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |3||1 | ||
| + | |- | ||
| + | | ||1 | ||
| + | |- | ||
| + | | ||7 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ג||א | ||
| + | |- | ||
| + | | ||א | ||
| + | |- | ||
| + | | ||ז | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | ::<span style=color:Green>[Illustration of the procedure:]</span> | ||
| + | ::{| | ||
| + | |- | ||
| + | | ||rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{Purple}{21\div7}}={\color{blue}{3}}}</math>|| <span style="color:#0000FF>3</span> ||rowspan="3"|<math>\scriptstyle\longrightarrow</math>||3 ||rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{Purple}{8\div7}}={\color{blue}{1}}+\frac{{\color{blue}{1}}}{7}}</math>||3<span style="color:#0000FF>1</span> | ||
| + | |- | ||
| + | |<span style="color:purple">21</span>8||218|| <span style="color:purple">8</span>|| <span style="color:#0000FF>1</span> | ||
| + | |- | ||
| + | | <span style="color:purple">7</span> || 7 || <span style="color:purple">7</span>|| 7 | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::We find that there are 31 times 7 in 218 and 1 remains. | ||
| + | |style="text-align:right;"|נמצא ברי"ח יש ל"א פעמי' ז' <s>וכן לעולם</s> וישאר א‫' | ||
| + | |- | ||
| + | | | ||
| + | ::And so on, examine it. | ||
| + | |style="text-align:right;"|וכן לעולם ועיין‫<ref>marg.: הג"ה דלעיל שייך ל[…]</ref> | ||
| + | |- | ||
| + | | | ||
| + | *Another example: I have 10127 pasin [?] and I want to know how many zehuvim are they, if each [zahuv] is 15 pasin? | ||
| + | :<math>\scriptstyle10127\div15</math> | ||
| + | |style="text-align:right;"|דומיון [אחר]‫<ref>M om.</ref> יש לי עשרת אלפים בצן ומאה ועשרים ושבע בצן ואבקש לידע כמה זהובי' בתוכו שכל אחד ט"ו פצן | ||
| + | |- | ||
| + | | | ||
| + | ::Write it like this: | ||
| + | |style="text-align:right;"|והנה רשמהו כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |1||0||1||2||7 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |א||0||א||ב||ז | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Write 15 beneath it: if you write it beneath the last ones, as required, we will find 1 beneath 1 and you will have nothing left. So, from where will you take 1 times 5? For, as the number of times you take one digit, so you should take the other, as I wrote above. Therefore, we shift 15 back and write the 1 beneath the zero and write 5 beneath 1 before the zero. It is like this: | ||
| + | |style="text-align:right;"|והנה תרשום תחת זה ה"א ואם תכתבנו תחת הסופיים כמחוייב נמצא א' תחת א' ולא ישאר לך דבר וא"כ מאין תקח אפ"ה כי כמה ‫<ref>114r</ref>פעמים שתקח אות אחת כמו כן תקח חבירתה כמו שכתבתי לעיל וא"כ נסוג [ה'א']‫<ref>M om.</ref> אחור ונכתוב הא' תחת [גלגל וכתו' ה' תחת א' שלפני גלגל ויהיה]‫<ref>M הא"0</ref> כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |1||0||1||2||7 | ||
| + | |- | ||
| + | | ||1||5|| || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |א||0||א||ב||ז | ||
| + | |- | ||
| + | | ||א||ה|| || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::We have to think that when we take the 1 from the digits above it, there should also be enough for the 5 in the remainder of this number: | ||
| + | |style="text-align:right;"|והנה צריכין אנו לשער באם נקח הא' מהאותיות שעליו שגם יעדיף למצא הה' בהעודף בחשבון הזה | ||
| + | |- | ||
| + | | | ||
| + | ::If you want to say: take 9 times from ten, then only 1 will remain. Add it instead of the zero; it is 11 and now you will not be able to take 9 times 5 from 11. | ||
| + | |style="text-align:right;"|ואם תרצה לומר ליקח ט' פעמי' מעשרה א"כ לא ישאר רק א' וחברו במקום הגלגל [ויהיה א"א]‫<ref>M om.</ref> ומעתה לא תוכל ליקח טפ"ה מא"א | ||
| + | |- | ||
| + | | | ||
| + | ::The same if you take 8 times 1, only 2 will remain and you will not be able to take 8 times 5 from 12. | ||
| + | |style="text-align:right;"|וכן אם תקח ח' פעמי' ב' מן א"ב [וכן אם תקח ח' פעמים א' לא ישאר רק ב' ולא תוכל ליקח ח' פעמים ה' מן ב"א]‫<ref>M om.</ref> | ||
| + | |- | ||
| + | | | ||
| + | ::Also if you take 7 times [1] from ten, only [3] will remain and you will not be able to take 7 times 5 from 31. | ||
| + | |style="text-align:right;"|וכן אם תקח <sup>ז"פ</sup> [מעשרה לא תוכל ליקח חפ"ה]<ref>marg.</ref> לא ישאר לך אלא א'ג' ולא תוכל ליקח ז' פעמים ה' מן א"ג | ||
| + | |- | ||
| + | | | ||
| + | ::But, take 6; 4 remains. | ||
| + | |style="text-align:right;"|אלא תקח ו' וישאר ד‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Write 6 above the 1 and it is like this: | ||
| + | |style="text-align:right;"|ואז כתוב [ו']‫<ref>M om.</ref> על הא' [ויהיה]‫<ref>M om.</ref> כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | | ||6|| || | ||
| + | |- | ||
| + | |4||1||2||7 | ||
| + | |- | ||
| + | | ||5|| || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | | ||ו|| || | ||
| + | |- | ||
| + | |ד||א||ב||ז | ||
| + | |- | ||
| + | | ||ה|| || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Then, you can take 6 times 5 from 41; you have 1 left from the 4, which is ten. Write 1 instead of the 4 and erase the 5. It is like this: | ||
| + | |style="text-align:right;"|ואז תוכל ליקח ו' פעמי' ה' מן א'ד' וישאר לך מן הד' שהיא א' עשרה ואח"כ כתוב א' במקום הד' [ומחק ה' ויהיה]‫<ref>M om.</ref> כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | | ||6|| || | ||
| + | |- | ||
| + | |1||1||2||7 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | | ||ו|| || | ||
| + | |- | ||
| + | |א||א||ב||ז | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | ::<span style=color:Green>[Illustration of the procedure:]</span> | ||
| + | ::{| | ||
| + | |- | ||
| + | | ||rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{Purple}{10-\left({\color{blue}{6}}\sdot1\right)}}={\color{blue}{4}}}</math>|| <span style="color:#0000FF>6</span>  ||rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{Purple}{40-\left(6\sdot5\right)}}={\color{blue}{1}}{\color{Purple}{0}}}</math>|| 6   | ||
| + | |- | ||
| + | |<span style="color:purple">10</span>127||<span style="color:#0000FF>4</span>127||<span style="color:#0000FF>1</span>127 | ||
| + | |- | ||
| + | | <span style="color:purple">1</span>5  || <span style="color:purple">5</span>   | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::We then want to take 1 from this number as follows: | ||
| + | |style="text-align:right;"|ואח"כ בקשנו ליקח הא' מן החשבון כזה | ||
| + | |- | ||
| + | | | ||
| + | ::You cannot take 10, as I explained. Apply this rule: you can never take 10, only 9, or 8, or 7, or less. | ||
| + | |style="text-align:right;"|י' פעמי' לא תוכל ליקח כמו שפירשתי וגם זה הכלל נקוט בידך לעולם לא תוכל ליקח י' רק ט' או ח' או ז' [ולמטה]‫<ref>M: וכו'</ref> | ||
| + | |- | ||
| + | | | ||
| + | ::So, do the following: write the 1 beneath the upper 12, like this: | ||
| + | |style="text-align:right;"|לכן עשה כך כתוב הא' תחת ב"א עליונה כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | | ||6|| || | ||
| + | |- | ||
| + | |1||1||2||7 | ||
| + | |- | ||
| + | | ||1||5|| | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | | ||ו|| || | ||
| + | |- | ||
| + | |א||א||ב||ז | ||
| + | |- | ||
| + | | ||א||ה|| | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::We take 7 times [1]; 4 remains, which is the excess of 11 over 7. | ||
| + | |style="text-align:right;"|ונקח ז' פעמי' נשאר ד' שעודף י"א על ז‫' | ||
| + | |- | ||
| + | | | ||
| + | ::We write 7 above, corresponding to the bottom 5, and to the upper 2. We erase the bottom 1. We erase also the upper 11 and write 4 beneath, like this: | ||
| + | |style="text-align:right;"|ונכתוב ז' למעלה כנגד הה' תחתונה ונמחק הא' תחתונה וכנגד ב' עליונה ונמחק ג"כ הא"א עליונה ונכתב תחתיהן ד' כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |6||7|| | ||
| + | |- | ||
| + | |4||2||7 | ||
| + | |- | ||
| + | | ||5|| | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ו||ז|| | ||
| + | |- | ||
| + | |ד||ב||ז | ||
| + | |- | ||
| + | | ||ה|| | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::We take 7 times 5 from the 4 next to it, i.e. 35; 4 remains, because the 4, which is in the second rank to 5, becomes forty. We take 35 from it; 5 remains. Add the 5 to the 2; it is 7, like this: | ||
| + | |style="text-align:right;"|והנה נקח ז' פעמי' ה' מן [ד']‫<ref>M om.</ref> שבצידה דהיינו ל"ה ויעדיף חמשה כי הד' היא במעלה שנייה מן הה' [עולה]‫<ref>M om.</ref> ארבעי' ועתה לקחנו ממנו ל"ה נשאר ה' צרוף הה' אל הב' שלפניה ויהיה ז' כזה | ||
| + | |- | ||
| + | | | ||
| + | ::15 is written beneath the remaining 77, like this: | ||
| + | |style="text-align:right;"|והנה נכתב ה'א' תחת ז'ז' העודף כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |6||7|| | ||
| + | |- | ||
| + | | ||7||7 | ||
| + | |- | ||
| + | | ||1||5 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ו||ז|| | ||
| + | |- | ||
| + | | ||ז||ז | ||
| + | |- | ||
| + | | ||א||ה | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | ::<span style=color:Green>[Illustration of the procedure:]</span> | ||
| + | ::{| | ||
| + | |- | ||
| + | | 6  ||rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{Purple}{11-\left({\color{blue}{7}}\sdot1\right)}}={\color{blue}{4}}}</math>||6<span style="color:#0000FF>7</span> ||rowspan="3"|<math>\scriptstyle\xrightarrow{\begin{cases}\scriptstyle{\color{Purple}{40-\left(7\sdot5\right)}}={\color{green}{5}}\\\scriptstyle{\color{Purple}{2+}}{\color{green}{5}}={\color{blue}{7}}\end{cases}}</math>||67  | ||
| + | |- | ||
| + | |<span style="color:purple>11</span>27||<span style="color:#0000FF>4</span>27|| <span style="color:#0000FF>7</span>7 | ||
| + | |- | ||
| + | | <span style="color:purple">1</span>5 || <span style="color:purple">5</span> || | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::We take 5 times 1 from 7, because we cannot take 7 times, as I explained above, since then we cannot take 7 times, or 6 times 5. So, take 5 times and write 5 above, next to the 7, corresponding to the bottom 5. 2 remains. Write the 2 instead of the 7, like this: | ||
| + | |style="text-align:right;"|ונקח ה' פעמי' א' מז' [כי ז' פעמים לא נוכל ליקח כמו שפי' לעיל שאז לא נוכל ליקח]‫<ref>M om.</ref> ז' פעמי' או ו' פעמי' ה' ודו"ק לכן ניקח ה' פעמי' ונכתב ה' למעל' בצד הז' [נגד ה' תחתונה]‫<ref>M om.</ref> ויעדיף ב' [אותו ב' כתוב במקום ז']‫<ref>M om.</ref> כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |6||7||5 | ||
| + | |- | ||
| + | | ||2||7 | ||
| + | |- | ||
| + | | || ||5 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ו||ז||ה | ||
| + | |- | ||
| + | | ||ב||ז | ||
| + | |- | ||
| + | | || ||ה | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::We take 5 times 5 from 27; 2 remains, which is less than the first number. The diagram is like this: | ||
| + | |style="text-align:right;"|והנה ניקח ה' פעמי' ה' מז"ב ויעדיף ב' שאינו מגיע <s>לחתן</s> לחשבון הא' וצורתו כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |6||7||5 | ||
| + | |- | ||
| + | | || ||2 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ו||ז||ה | ||
| + | |- | ||
| + | | || ||ב | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::We find correctly that ten thousand, one hundred and 27 pasin [?] are 6[7]5 zehuvim and two pasin [?]. | ||
| + | |style="text-align:right;"|נמצא מכוון שעשרת אלפי' ומאה וכ"ז [פצין עולה ה' זהו' וגם ת"ר זהו' ושני פצין]‫<ref>M: בצן ו ע"ה ות"ר זהו' ושני בצן</ref> | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | ::<span style=color:Green>[Illustration of the procedure:]</span> | ||
| + | ::{| | ||
| + | |- | ||
| + | |67 ||rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{Purple}{7-\left({\color{blue}{5}}\sdot1\right)}}={\color{blue}{2}}}</math>||67<span style="color:#0000FF>5</span>||rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{Purple}{27-\left(5\sdot5\right)}}={\color{blue}{2}}}</math>||675 | ||
| + | |- | ||
| + | | <span style="color:purple">7</span>7|| <span style="color:#0000FF>2</span>7||  <span style="color:#0000FF>2</span> | ||
| + | |- | ||
| + | | <span style="color:purple">1</span>5||  <span style="color:purple">5</span>|| 15 | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | === <span style=color:green>Checking Methods</span> === | ||
| + | | | ||
| + | |- | ||
| + | |If you have doubts multiply the last [result] by 15 and you will receive the original number you asked about. | ||
| + | |style="text-align:right;"|ואם לבך מגמגם כפול העודף האחרון על ה'א' ויצא לך חשבון הראשון ששאלת | ||
| + | |- | ||
| + | |This is the scales of the division chapter. | ||
| + | |style="text-align:right;"|וזהו מאזני צדק על שער החילוק | ||
| + | |- | ||
| + | |We find that the multiplication is verified by division and the division is verified by multiplication. | ||
| + | |style="text-align:right;"|נמצא כפל מבורר החילוק והחילוק מברר הכפל | ||
| + | |- | ||
| + | | | ||
| + | *Example for scales: | ||
| + | :<math>\scriptstyle15\times\left(675+\frac{2}{15}\right)\longrightarrow10127\div15</math> | ||
| + | |style="text-align:right;"|דמיון למאזנים | ||
| + | |- | ||
| + | | | ||
| + | ::Write 15 and beneath it write [67]5, which is the recent remainder, except for the 2 that is less than 15, which you will add at the end. Like this: | ||
| + | |style="text-align:right;"|כתוב ה'א' וכתוב תחתיו הו"ז הנשאר באחרונה חוץ מהב' שלא הגיע לחשבון ט"ו אל תצטרף עמהם עד סופו חברה לאות ראשונה כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | | || ||1||5 | ||
| + | |- | ||
| + | |6||7||5|| | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | | || ||א||ה | ||
| + | |- | ||
| + | |ו||ז||ה|| | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Multiply 6 by 1; the result is 6. | ||
| + | |style="text-align:right;"|כפול ו' על א' יעלה ו‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Multiply 7 by 1; the result is 7. | ||
| + | |style="text-align:right;"|כפול ז' על א' יעלה ז‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Multiply 5 by 1; the result is 5. Like this: | ||
| + | |style="text-align:right;"|כפול ‫<ref>114v</ref>ה' על א' יעלה ה' כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |6||7||5||5 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ו||ז||ה||ה | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Write 675 beneath the 5 of 15, like this: | ||
| + | |style="text-align:right;"|והנה כתוב הז"ו תחת ה' של ה"א כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |6||7||5||5 | ||
| + | |- | ||
| + | | ||6||7||5 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ו||ז||ה||ה | ||
| + | |- | ||
| + | | ||ו||ז||ה | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Multiply 6 by 5; the result is 30. | ||
| + | |style="text-align:right;"|כפול ו' על ה' יעלה ל‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Add it to the [6] above it, as I taught you in the multiplication chapter: so, you need to add the 3 to the 6; it is 9. Like this: | ||
| + | |style="text-align:right;"|חבריהו לז' שעליו כמו שציויתיך בשער הכפל א"כ צריך אתה ליתן הג' אל הו' ויהיה ט' כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |9||7||5||5 | ||
| + | |- | ||
| + | | || ||7||5 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ט||ז||ה||ה | ||
| + | |- | ||
| + | | || ||ז||ה | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Then, multiply 7 by 5; the result is 35; plus 5; it is 40. | ||
| + | |style="text-align:right;"|ואח"כ כפול ז' על ה' ויעלה ה"ג וה' דהיינו 0"ד | ||
| + | |- | ||
| + | | | ||
| + | ::Write zero instead of the 5 and add 4 to 7; it is 11. Write 1 instead of the 7 and add 1 to the 9; it is 10. So, you have to write a zero instead of the 9 and 1 after it. Like this: | ||
| + | |style="text-align:right;"|עשה גלגל במקום ה' ותן ד' לז' ויהיה א'א' כתוב א' במקום ז' ותן א' אל הט' ויהיה י' אז צריך אתה לעשות במקום הט' גלגל ואחריו א' כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |1||0||1||0||5 | ||
| + | |- | ||
| + | | || || || ||5 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |א||0||א||0||ה | ||
| + | |- | ||
| + | | || || || ||ה | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Next, multiply 5 by 5; the result is 25. | ||
| + | |style="text-align:right;"|ואח"כ כפול ה' על ה' ויעלה ה"ב | ||
| + | |- | ||
| + | | | ||
| + | ::Write 5 instead of the upper 5, but do not add it to the first 5 above, as I taught you in the multiplication chapter. Then, write 2 instead of the zero, like this: | ||
| + | |style="text-align:right;"|כתוב ה' במקום ה' העליונה ואל תצרף ה' ראשונ' עליה כמו שציויתיך בשער הכפל ודו"ק ואח"כ כתוב ב' במקום גלגל כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |style="text-align:right;"|1||0||1||2||5 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |style="text-align:right;"|א||0||א||ב||ה | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Add the 2 that is less than 15 to the first 5; it is 7. So, the number is like this: | ||
| + | |style="text-align:right;"|והנה חבר ב' שלא הגיע לכלל ט"ו אל הה' הראשונה ויהיה ז' ואז יהיה החשבון כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |style="text-align:right;"|1||0||1||2||7 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |style="text-align:right;"|א||0||א||ב||ז | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::So, the question is verified. | ||
| + | |style="text-align:right;"|והיינו כיון השאלה | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | ::<span style=color:Green>[Illustration of the procedure:]</span> | ||
| + | ::{| | ||
| + | |- | ||
| + | |  <span style="color:purple">1</span>5||rowspan="2"|<math>\scriptstyle\xrightarrow{\begin{cases}\scriptstyle{\color{Purple}{1\times6}}={\color{blue}{6}}\\\scriptstyle{\color{Purple}{1\times7}}={\color{blue}{7}}\\\scriptstyle{\color{Purple}{1\times5}}={\color{blue}{5}}\end{cases}}</math>||<span style="color:#0000FF>675</span><span style="color:purple">5</span>||rowspan="2"|<math>\scriptstyle\xrightarrow{\begin{cases}\scriptstyle{\color{Purple}{5\times6}}={\color{green}{3}}{\color{blue}{0}}\\\scriptstyle{\color{Purple}{6+}}{\color{green}{3}}={\color{blue}{9}}\end{cases}}</math>||<span style="color:#0000FF>9</span>75<span style="color:purple">5</span>||rowspan="2"|<math>\scriptstyle\xrightarrow{\begin{cases}\scriptstyle{\color{Purple}{5\times7}}={\color{green}{35}}\\\scriptstyle{\color{Purple}{5+}}{\color{green}{35}}={\color{green}{4}}{\color{blue}{0}}\\\scriptstyle{\color{Purple}{7+}}{\color{green}{4}}={\color{green}{1}}{\color{blue}{1}}\\\scriptstyle{\color{Purple}{9+}}{\color{green}{1}}={\color{blue}{10}}\end{cases}}</math>||<span style="color:#0000FF>1010</span><span style="color:purple">5</span> | ||
| + | |- | ||
| + | |<span style="color:purple">675</span> || <span style="color:purple">6</span>75||  <span style="color:purple">7</span>5||    <span style="color:purple">5</span> | ||
| + | |} | ||
| + | ::::::{| | ||
| + | |- | ||
| + | |<math>\scriptstyle\xrightarrow{\scriptstyle{\color{Purple}{5\times5}}={\color{blue}{25}}}</math>||101<span style="color:#0000FF>25</span>||<math>\scriptstyle\xrightarrow{\scriptstyle{\color{Purple}{5+2}}={\color{blue}{7}}}</math>||1012<span style="color:#0000FF>7</span> | ||
| + | |} | ||
| + | |- | ||
| + | |With God's help, the chapter of division is complete. | ||
| + | |style="text-align:right;"|בע"ה סליק שער החילוק | ||
| + | |- | ||
| + | | | ||
| + | *I will show you another example: I multiplied 568 times 568, like this: | ||
| + | ::<math>\scriptstyle568\times568</math> | ||
| + | |style="text-align:right;"|‫<ref>MS Paris1088, 5r-v</ref>ועוד אראה לך דומיון חושבתי מן חו"ה פעמי' חו"ה הכי | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |3||2||2||6||2||4 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ג||ב||ב||ו||ב||ד | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::I want to divide, [in order to check] if I multiplied correctly. Do as follows: | ||
| + | ::<math>\scriptstyle322624\div568</math> | ||
| + | |style="text-align:right;"|ורציתי לחלק אם חושבתי שפיר וכה תעשה | ||
| + | |- | ||
| + | | | ||
| + | ::Write the result and write 568 beneath it. Its diagram is like this: | ||
| + | |style="text-align:right;"|תכתוב לפניך החשבון שעולה ממנה ותכתוב תחתיו חו"ה וצורתו כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |3||2||2||6||2||4 | ||
| + | |- | ||
| + | | ||5||6||8|| || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ג||ב||ב||ו||ב||ד | ||
| + | |- | ||
| + | | ||ה||ו||ח|| || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Since you cannot divide 3 by 5, because 3 does not reach 5 even once, shift 5 backwards. | ||
| + | |style="text-align:right;"|כי לא אתה יכול לחלק ה' מן ג' כי ג' לא מגיע אפי' פעם אחת לה' לפיכך סוג הה' לאחרי‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Then, say: how many times 5 is in 32? 5 times, although I can take one more time. | ||
| + | |style="text-align:right;"|ואז תאמר כמה פעמים ה' בב"ג זהו ה' פעמים אע"ג שיש לי לקח עוד פעם אחת ה‫' | ||
| + | |- | ||
| + | | | ||
| + | ::The reason I take it 5 times, not more, is that if I take 6 times, only 2 will remain from 32. | ||
| + | ::<math>\scriptstyle{\color{blue}{32-\left(5\sdot6\right)=2}}</math> | ||
| + | |style="text-align:right;"|אלא מטעם זה אני לקח נוטל אות' ה' פעמים ולא יותר כי אם אני נוטל ו' פעמי' אז לא היה נשאר מן הב"ג אלא ב‫' | ||
| + | |- | ||
| + | | | ||
| + | ::But, then it will be impossible to take also 6 times the 6 of 568 from 22. | ||
| + | ::<math>\scriptstyle{\color{blue}{22-\left(6\sdot6\right)=\varnothing}}</math> | ||
| + | |style="text-align:right;"|ואז לא היה יכול לקח הו' מן חו"ה נמי ו' פעמי' מן ב"ב פי' מן כ"ב | ||
| + | |- | ||
| + | | | ||
| + | ::The rule in this chapter is that as many times you divide the first digit, so you do with all other digits. | ||
| + | |style="text-align:right;"|והדין בזה השער כי כמה פעמי' שתחלק האות ראשו' אז אתה עושה לכל האותיות | ||
| + | |- | ||
| + | | | ||
| + | ::Therefore, I take 5 times, so 7 remains and then I can take the 6 also 5 times. | ||
| + | ::<math>\scriptstyle{\color{blue}{32-\left(5\sdot5\right)=7}}</math> | ||
| + | |style="text-align:right;"|לפי זה אני נוטל ה' פעמי' ואז נשאר ז' ואז אני יכול לנטול גם הו' ה' פעמים | ||
| + | |- | ||
| + | | | ||
| + | ::Write the 5 above the 6, corresponding to the lower 8. | ||
| + | |style="text-align:right;"|ואות' ה' תכתוב למעלה על הו' נגד הח' התחתונ‫' | ||
| + | |- | ||
| + | | | ||
| + | ::We erase the 32 and write the 7 instead. This is its diagram: | ||
| + | |style="text-align:right;"|ונמחוק הב"ג ותכתוב במקומ' הז' וצורתו כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | | || ||5|| || | ||
| + | |- | ||
| + | |7||2||6||2||4 | ||
| + | |- | ||
| + | | ||6||8|| || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | | || ||ה|| || | ||
| + | |- | ||
| + | |ז||ב||ו||ב||ד | ||
| + | |- | ||
| + | | ||ו||ח|| || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Again, [subtract] the 6 of 568 also 5 times from 72; you are left with 42. | ||
| + | ::<math>\scriptstyle{\color{blue}{72-\left(6\sdot5\right)=42}}</math> | ||
| + | |style="text-align:right;"|ושוב תחלוק הו' מן חו"ה נמי ה' פעמים מן ב"ז ואז נשאר לך ב"ד | ||
| + | |- | ||
| + | | | ||
| + | ::Erase the 7 and write 4 instead. This is its diagram: | ||
| + | |style="text-align:right;"|ותמחוק הז' ותכתוב במקו' ד' וצורתו כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | | || ||5|| || | ||
| + | |- | ||
| + | |4||2||6||2||4 | ||
| + | |- | ||
| + | | || ||8|| || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | | || ||ה|| || | ||
| + | |- | ||
| + | |ד||ב||ו||ב||ד | ||
| + | |- | ||
| + | | || ||ח|| || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::[Subtract] the 8 also 5 times from 426, meaning 4 hundred and 26: take a hundred from the 4 hundred; 3 hundred remain. | ||
| + | ::<math>\scriptstyle{\color{blue}{400-100=300}}</math> | ||
| + | |style="text-align:right;"|ואז תחלוק הח' נמי ה' פעמי' מן וב"ד פי' כ"ו ד' מאות אז תקח מאה מן הד' מאו' ועוד נשאר ג' מאות | ||
| + | |- | ||
| + | | | ||
| + | ::Then, take the 8 times 5, which is 40, from the hundred; 60 remains. | ||
| + | ::<math>\scriptstyle{\color{blue}{100-\left(8\sdot5\right)=100-40=60}}</math> | ||
| + | |style="text-align:right;"|ואז קח מן המאה הח' פעם ה' וזהו 0"ד פי' מ' ועוד נשאר 0"ו | ||
| + | |- | ||
| + | | | ||
| + | ::Add the 6 to the 2; it is 8 and this is its diagram: | ||
| + | ::<math>\scriptstyle{\color{blue}{6+2=8}}</math> | ||
| + | |style="text-align:right;"|ואותו ו' תשים על הב' ויהיה ח' וצורתו כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | | || ||5|| || | ||
| + | |- | ||
| + | |3||8||6||2||4 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | | || ||ה|| || | ||
| + | |- | ||
| + | |ג||ח||ו||ב||ד | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | ::<span style=color:Green>[Illustration of the procedure:]</span> | ||
| + | ::{| | ||
| + | |- | ||
| + | | ||rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{Purple}{32-\left({\color{blue}{5}}\sdot5\right)}}={\color{blue}{7}}}</math>||  <span style="color:#0000FF>5</span>  ||rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{Purple}{72-\left(5\sdot6\right)}}={\color{blue}{42}}}</math>||  5  ||rowspan="3"|<math>\scriptstyle\xrightarrow{\begin{cases}\scriptstyle{\color{Purple}{400-100}}={\color{blue}{3}}{\color{Purple}{00}}\\\scriptstyle{\color{Purple}{100-\left(5\sdot8\right)}}={\color{green}{6}}{\color{Purple}{0}}\\\scriptstyle{\color{Purple}{2+}}{\color{green}{6}}={\color{blue}{8}}\end{cases}}</math>||  5   | ||
| + | |- | ||
| + | |<span style="color:purple">32</span>2624||<span style="color:#0000FF>7</span>2624||<span style="color:#0000FF>42</span>624||<span style="color:#0000FF>38</span>624 | ||
| + | |- | ||
| + | | <span style="color:purple">5</span>68 || <span style="color:purple">6</span>8  ||  <span style="color:purple">8</span>  || | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::We shift 568 backwards beneath the 2; this is its diagram: | ||
| + | |style="text-align:right;"|נמשוך חו"ה לאחריה תחת הב' וצורתו כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | | || ||5|| || | ||
| + | |- | ||
| + | |3||8||6||2||4 | ||
| + | |- | ||
| + | | ||5||6||8|| | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | | || ||ה|| || | ||
| + | |- | ||
| + | |ג||ח||ו||ב||ד | ||
| + | |- | ||
| + | | ||ה||ו||ח|| | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Then, say: how many times 5 is in 38; the result is 6 and 8 remains. | ||
| + | ::<math>\scriptstyle{\color{blue}{38-\left(5\sdot6\right)=8}}</math> | ||
| + | |style="text-align:right;"|ואז תאמר כמה פעמי' ה' בח"ג ויעלה ו' ועוד נשאר ח‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Erase the 3 that follows the 8 and write the 6 resulting from the 38 above the 2 in the upper line, corresponding to the lower 8. This is its diagram: | ||
| + | |style="text-align:right;"|ותמחוק הג' שאחר הח' והו' העולה מן הח"ג תכתו' על הב' בשור' עליונ' נגד הח' שלמטה וצורתו כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | | ||5||6|| | ||
| + | |- | ||
| + | |8||6||2||4 | ||
| + | |- | ||
| + | | ||6||8|| | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | | ||ה||ו|| | ||
| + | |- | ||
| + | |ח||ו||ב||ד | ||
| + | |- | ||
| + | | ||ו||ח|| | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Take the 6 also 6 times from 86 and do as follows: take 3[6] from 8[6]; 50 remains. Take the 6 before the 8 and write a zero instead, because 6 times 6 is 36 and this is its diagram: | ||
| + | ::<math>\scriptstyle{\color{blue}{86-\left(6\sdot6\right)=86-36=50}}</math> | ||
| + | |style="text-align:right;"|ואז תקח הו' נמי ו' פעמי' מן ו"ח פי' מן פ"ו ועשה הכי תקח ה"ג פי' ה"ל מן ה"ח ואז נשאר נ' וקח הו' שלפני הח' ושים במקומ' גלגל כי ו' פעמי' ו' זהו ו"ג וצורתו כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | | ||5||6|| | ||
| + | |- | ||
| + | |5||0||2||4 | ||
| + | |- | ||
| + | | || ||8|| | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | | ||ה||ו|| | ||
| + | |- | ||
| + | |ה||0||ב||ד | ||
| + | |- | ||
| + | | || ||ח|| | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Erase the 6 of 568, as I wrote. | ||
| + | |style="text-align:right;"|ותמחוק הו' של חו"ה כמו שציירתי | ||
| + | |- | ||
| + | | | ||
| + | ::Take again the 8 also 6 times from 5 hundred. Do this as follows: take a hundreds from the 5 hundred; 4 hundred remain. | ||
| + | ::<math>\scriptstyle{\color{blue}{500-100=400}}</math> | ||
| + | |style="text-align:right;"|ושוב קח הח' נמי ו' פעמים מן ה' מאות ואז שוב עשה הכי קח מאה מן הה' מאות ועוד נשאר ד' מאות | ||
| + | |- | ||
| + | | | ||
| + | ::Take 8 times 6, which is 48 from the hundred: take 50 from the hundred; 50 remains. Write the 50 instead of the zero before the 4. | ||
| + | ::<math>\scriptstyle{\color{blue}{100-50=50}}</math> | ||
| + | |style="text-align:right;"|וקח מן המאה ח' פעמי' ו' וזהו ח"ד פי' מ"ח ואז קח מן המאה נ' ואז נשאר נ' ואות' נ' תכתו' במקו' הגלגל שלפני הד‫' | ||
| + | |- | ||
| + | | | ||
| + | ::I still need 2 for the 48 I take from 50. Add the 2 to the 2 after the first 4. | ||
| + | ::<math>\scriptstyle{\color{blue}{50-\left(8\sdot6\right)=50-48=2}}</math> | ||
| + | |style="text-align:right;"|ועוד יש ב' יותר שאני צריך אלא מ"ח ואני לקחתי נ' ואות' ב' תשים על הב' של אחר הד' הראשונ‫' | ||
| + | |- | ||
| + | | | ||
| + | ::We erase the 8 from the 568. This is its diagram: | ||
| + | |style="text-align:right;"|ונמחוק הח' מן חו"ה וצורתו כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | | ||5||6|| | ||
| + | |- | ||
| + | |4||5||4||4 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | | ||ה||ו|| | ||
| + | |- | ||
| + | |ד||ה||ד||ד | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | ::<span style=color:Green>[Illustration of the procedure:]</span> | ||
| + | ::{| | ||
| + | |- | ||
| + | |  5  ||rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{Purple}{38-\left({\color{blue}{6}}\sdot5\right)}}={\color{blue}{8}}}</math>|| 5<span style="color:#0000FF>6</span> ||rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{Purple}{86-\left(6\sdot6\right)}}={\color{blue}{50}}}</math>|| 56 ||rowspan="3"|<math>\scriptstyle\xrightarrow{\begin{cases}\scriptstyle{\color{Purple}{500-100}}={\color{blue}{4}}{\color{Purple}{00}}\\\scriptstyle{\color{Purple}{100-}}{\color{green}{50}}={\color{blue}{5}}{\color{Purple}{0}}\\\scriptstyle{\color{green}{50-}}{\color{Purple}{\left(6\sdot8\right)}}={\color{green}{2}}{\color{Purple}{0}}\\\scriptstyle{\color{Purple}{2+}}{\color{green}{2}}={\color{blue}{4}}\end{cases}}</math>|| 56  | ||
| + | |- | ||
| + | |<span style="color:purple">38</span>624||<span style="color:#0000FF>8</span>624||<span style="color:#0000FF>50</span>24||<span style="color:#0000FF>454</span>4 | ||
| + | |- | ||
| + | | <span style="color:purple">5</span>68 || <span style="color:purple">6</span>8 ||  <span style="color:purple">8</span> || | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::We shift 568 backwards: the 8 beneath the 4, the 6 beneath the second 4, and the 5 beneath the 5. This is its diagram: | ||
| + | |style="text-align:right;"|נמשוך חו"ה לאחריה תח הח' תחת הד' והו' תחת הד' השניי' והה' תחת הה' וצורתו כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | | ||5||6|| | ||
| + | |- | ||
| + | |4||5||4||4 | ||
| + | |- | ||
| + | | ||5||6||8 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | | ||ה||ו|| | ||
| + | |- | ||
| + | |ד||ה||ד||ד | ||
| + | |- | ||
| + | | ||ה||ו||ח | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Then, say: how many times 5 is in 45; the result is 8 times. Write the 8 above the 4, corresponding to the lower 8. 5 remains. | ||
| + | ::<math>\scriptstyle{\color{blue}{45-\left(5\sdot8\right)=5}}</math> | ||
| + | |style="text-align:right;"|ואז תאמר כמה פעמי' ה' בה"ד פי' מ"ה ויעלה ח' פעמי' ואות' ח' תכתו' למעלה על הד' נגד ח' התחתונ' ועוד נשאר ה‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Erase the 4 after the 5 and erase the 5 of 568. This is its diagram: | ||
| + | |style="text-align:right;"|ותמחוק הד' שאחר הה' ותמחוק הה' של חו"ה וצורתו כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |5||6||8 | ||
| + | |- | ||
| + | |5||4||4 | ||
| + | |- | ||
| + | | ||6||8 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ה||ו||ח | ||
| + | |- | ||
| + | |ה||ד||ד | ||
| + | |- | ||
| + | | ||ו||ח | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::We [subtract] again the 6 also 8 times from 54. Do it as follows: take 8 times 6 from the 50; 2 remains, because 8 times 6 is 48, so 2 remains. | ||
| + | ::<math>\scriptstyle{\color{blue}{50-\left(6\sdot8\right)=50-48=2}}</math> | ||
| + | |style="text-align:right;"|ושוב נחלק הו' נמי ח' פעמים מן ד"ה פי' מן נ"ה וע ועשה הכי קח מן הנ' ח' פעמי' ו' ועוד נשאר ב' כי ח' פעמי' ו' זהו ח"ד פי' מ"ח ועוד נשאר ב‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Add the 2 to the 4 before the 5; the result is 6. | ||
| + | ::<math>\scriptstyle{\color{blue}{2+4=6}}</math> | ||
| + | |style="text-align:right;"|ואות' ב' תשים על הד' שלפני הה' והוה ו' וצור | ||
| + | |- | ||
| + | | | ||
| + | ::We erase the 6 of 568 and the 5 that follows the 4. This is the diagram: | ||
| + | |style="text-align:right;"|ונמחק ו' של חו"ה והה' אחר הד' וצורת' כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |5||6||8 | ||
| + | |- | ||
| + | | ||6||4 | ||
| + | |- | ||
| + | | || ||8 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ה||ו||ח | ||
| + | |- | ||
| + | | ||ו||ד | ||
| + | |- | ||
| + | | || ||ח | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Then, say: 8 times 8 is 64. | ||
| + | ::<math>\scriptstyle{\color{blue}{8\sdot8=64}}</math> | ||
| + | |style="text-align:right;"|ואז תאמר ח' פעמי' ח' וזהו ד"ו פי' ס"ד | ||
| + | |- | ||
| + | | | ||
| + | ::The example of the chapter on division is complete. | ||
| + | |style="text-align:right;"|סליק דומיון של שער החילוק | ||
| + | |- | ||
| + | | | ||
| + | ::We find that when I divide 322624 by 568 nothing remains. | ||
| + | |style="text-align:right;"|נמצא כשחלקתי חו"ה מן ד'ב'ו'ב'ב'ג' אז לא נשאר מאומה | ||
| + | |- | ||
| + | |Likewise, whenever you multiply by the same thing, as 568 times 568, or 464 times 464, then you divide by what you multiplied and you have nothing left, the calculation is correct. | ||
| + | |style="text-align:right;"|וכן בכל מקום אשר אתה מחשב דבר השוה כגו' ח'ו'ה' פעמי' ח'ו'ה' או דו"ד פעמי' דו"ד וכשתחלק ממנ' אות' שחשבת ולא נשאר לך מאומה אז החשבון אמת ודו"ק ותמצא | ||
| + | |- | ||
| + | | | ||
| + | *I will show you another example, in which the product is not by the same, as 21 times 45: | ||
| + | ::<math>\scriptstyle21\times45</math> | ||
| + | |style="text-align:right;"|ועוד אראה לך דומיון שאינ' חשבון שוה כגו' א"ב פעמי' ה"ד | ||
| + | |- | ||
| + | | | ||
| + | ::Then you do not use this way, but take one of the multiplicands, the 21 or the 45. Divide the product by it and the result is the other multiplicand. | ||
| + | |style="text-align:right;"|ואז אתה לא עושה כדרך זה קח חד מן הפרטות הא"ב או הה"ד ותחלק מן החשבון ואז עולה הפרט השיני ודו"ק | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | ::<span style=color:Green>[Illustration of the procedure:]</span> | ||
| + | ::{| | ||
| + | |- | ||
| + | | 56 ||rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{Purple}{45-\left({\color{blue}{8}}\sdot5\right)}}={\color{blue}{5}}}</math>||56<span style="color:#0000FF>8</span>||rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{Purple}{54-\left(8\sdot6\right)}}={\color{blue}{6}}}</math>||568||rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{Purple}{64-\left(8\sdot8\right)}}={\color{blue}{0}}}</math>||568 | ||
| + | |- | ||
| + | |<span style="color:purple">45</span>44||<span style="color:#0000FF>5</span>44|| <span style="color:#0000FF>6</span>4|| | ||
| + | |- | ||
| + | | <span style="color:purple">5</span>68|| <span style="color:purple">6</span>8||  <span style="color:purple">8</span>|| | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | *Example: we multiplied 45 times 21 and the result is like this: | ||
| + | :<math>\scriptstyle45\times21\longrightarrow945\div45</math> | ||
| + | |style="text-align:right;"|‫<ref>MS Paris1088, 6r</ref>דומיון חשבנו ה"ד פעמים א"ב ועולה ממנ' כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |9||4||5 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ט||ד||ה | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | :So, we divide [to check] if we multiplied correctly: | ||
| + | |style="text-align:right;"|ואז נחלק אם חשבנו אמת | ||
| + | |- | ||
| + | | | ||
| + | :Take the resulting product and write one multiplicand beneath it. Say: how many times this multiplicand is in this product? The result is the other multiplicand. | ||
| + | |style="text-align:right;"|אז קח הכלל העולה ותכתוב תחתיו חד פרט ותאמר כמה פעם זה הפרט בזה הכלל אז עולה הפרט השני‫' | ||
| + | |- | ||
| + | | | ||
| + | :Here is the diagram, in which you write the product and beneath it one multiplicand, whichever you want: | ||
| + | |style="text-align:right;"|והילך צורתו אשר תכתביהו הכלל ותחתיו חד פרט איזה שתרצה | ||
| + | |- | ||
| + | | | ||
| + | ::Take the product 945 and write the multiplicand 45 beneath it. The diagram is like this: | ||
| + | |style="text-align:right;"|ועת' נרא' קח הכלל ה'ד'ט' ותכתוב תחתיו הפרט ה"ד וצורתו כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |9||4||5 | ||
| + | |- | ||
| + | |4||5|| | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ט||ד||ה | ||
| + | |- | ||
| + | |ד||ה|| | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Say: how many times 4 is in 9? We find 2 times, no more. | ||
| + | |style="text-align:right;"|ותאמר כמה פעמים ד' בט' נמצא ב' פעמי' ולא יותר | ||
| + | |- | ||
| + | | | ||
| + | ::Write the 2 above the 4, corresponding to the lower 5. | ||
| + | |style="text-align:right;"|אות' ב' תכתוב על הד' נגד הה' שלמט‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Erase the 9 and write 1 instead. | ||
| + | ::<math>\scriptstyle{\color{OliveGreen}{9-\left(4\sdot2\right)=1}}</math> | ||
| + | |style="text-align:right;"|ותמחוק הט' ותכתוב במקומ' א‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Erase the lower 4. The diagram is like this: | ||
| + | |style="text-align:right;"|<s>ואז קח</s> ותמחוק ד' תחתונ' וצורת' כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | | ||2|| | ||
| + | |- | ||
| + | |1||4||5 | ||
| + | |- | ||
| + | | ||5|| | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | | ||ב|| | ||
| + | |- | ||
| + | |א||ד||ה | ||
| + | |- | ||
| + | | ||ה|| | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Take the 5 also 2 times from 14; 4 remains. | ||
| + | ::<math>\scriptstyle{\color{blue}{14-\left(5\sdot2\right)=4}}</math> | ||
| + | |style="text-align:right;"|ואז קח הה' נמי ב' פעמי' מן ד"א ונשאר הד‫' | ||
| + | |- | ||
| + | | | ||
| + | ::We erase the lower 1 and 5. The diagram is like this: | ||
| + | |style="text-align:right;"|ונמחוק הא' והה' שלמט' וצורתו כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |2|| | ||
| + | |- | ||
| + | |4||5 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ב|| | ||
| + | |- | ||
| + | |ד||ה | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | ::<span style=color:Green>[Illustration of the procedure:]</span> | ||
| + | ::{| | ||
| + | |- | ||
| + | | ||rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{Purple}{9-\left({\color{blue}{2}}\sdot4\right)}}={\color{blue}{1}}}</math>|| <span style="color:#0000FF>2</span> ||rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{Purple}{14-\left(2\sdot5\right)}}={\color{blue}{4}}}</math>||2  | ||
| + | |- | ||
| + | |<span style="color:purple">9</span>45||<span style="color:#0000FF>1</span>45||<span style="color:#0000FF>4</span>5 | ||
| + | |- | ||
| + | |<span style="color:purple">4</span>5 || <span style="color:purple">5</span> || | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Shift the 45 one rank backwards: the 5 beneath the 5, and the 4 beneath the 4. The diagram is like this: | ||
| + | |style="text-align:right;"|ואז תמשוך הה"ד מעלה אחת לאחריה הה' תחת הה' והד' תחת הד' וצורתו כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |2|| | ||
| + | |- | ||
| + | |4||5 | ||
| + | |- | ||
| + | |4||5 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ב|| | ||
| + | |- | ||
| + | |ד||ה | ||
| + | |- | ||
| + | |ד||ה | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Say: how many times 4 is in 4? we find only 1. | ||
| + | ::<math>\scriptstyle{\color{blue}{4-\left(4\sdot1\right)=0}}</math> | ||
| + | |style="text-align:right;"|ואז תאמר כמה פעמי' ד' בד' נמצא רק א‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Write the 1 above the two 5's before the 2 and erase the second 4. The diagram is like this: | ||
| + | |style="text-align:right;"|ואות' א' תכתו' על השני ה' קודם הב' וצורתו כזה ונמחק השני ד' כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |2||1 | ||
| + | |- | ||
| + | | ||5 | ||
| + | |- | ||
| + | | ||5 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ב||א | ||
| + | |- | ||
| + | | ||ה | ||
| + | |- | ||
| + | | ||ה | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Then, take also the 5 once; you have nothing left. | ||
| + | |style="text-align:right;"|ואז <s>תאמר</s> תקח הה' נמי א' פעם אז לא נשאר לך מאומה | ||
| + | |- | ||
| + | | | ||
| + | :We find that when I divide by one multiplicand, the result is the other multiplicand. | ||
| + | |style="text-align:right;"|נמצא כשחלקתי חד פרט אז עולה הפרט השיני | ||
| + | |- | ||
| + | | | ||
| + | :You will find it so every time you divide. | ||
| + | |style="text-align:right;"|וכן תמצא בכל פעם אשר אתה מחלק | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | ::<span style=color:Green>[Illustration of the procedure:]</span> | ||
| + | ::{| | ||
| + | |- | ||
| + | |2 ||rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{Purple}{4-\left({\color{blue}{1}}\sdot4\right)}}={\color{blue}{0}}}</math>||2<span style="color:#0000FF>1</span>||rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{Purple}{5-\left(1\sdot5\right)}}={\color{blue}{0}}}</math>||21 | ||
| + | |- | ||
| + | |<span style="color:purple">4</span>5|| <span style="color:purple">5</span>|| | ||
| + | |- | ||
| + | |<span style="color:purple">4</span>5|| <span style="color:purple">5</span>|| | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | *Here is a good chapter to see if I divided well and correctly; I will show you an example: | ||
| + | :I want to see how many times 7 is in 2 hundred and 18. | ||
| + | :Since it is already divided at the beginning of the division chapter, the result is 31 and 1 remains. | ||
| + | :See if I divided correctly: | ||
| + | :<math>\scriptstyle218\div7\longrightarrow\left(31+\frac{1}{7}\right)\times7</math> | ||
| + | |style="text-align:right;"|‫<ref>MS Paris1088, 6r</ref>והילך שער טוב לראות אם חלקתי אמת וטוב מאד מאד ואראה לך דומיון<br> | ||
| + | רציתי לחלק כמה פעם ז' בב' מאות וי"ח<br> | ||
| + | כאשר נחלק כבר בתחילת שער החילוק והוה עולה [א"ג] ונשאר א' ואז ראה אם חלקתי אמת | ||
| + | |- | ||
| + | | | ||
| + | ::Take the 7, by which I divided, and write it beneath the 3, like this: | ||
| + | |style="text-align:right;"|קח הז' שחלקתי ושים אותה תחת הג' [‫כזה‫] | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |3||1 | ||
| + | |- | ||
| + | |7|| | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ג||א | ||
| + | |- | ||
| + | |ז|| | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Say: how much is 7 times 3? It is 21. | ||
| + | |style="text-align:right;"|ותאמר כמה ז' פעמים ג' זהו כ"א א"ב פי' כ"א | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |2||1||1 | ||
| + | |- | ||
| + | | || ||7 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ב||א||א | ||
| + | |- | ||
| + | | || ||ז | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Take the 7 and write it beneath the 1. | ||
| + | |style="text-align:right;"|ואז קח הז' ושים אות' תחת הא‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Say: 7 times 1 is 7. | ||
| + | |style="text-align:right;"|ותאמ' ז' פעמי' א' זהו ז‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Write 7 before the 21, which is the result of 3 times 6; like this: | ||
| + | |style="text-align:right;"|ואות' ז' תכתו' קודם הא"ב שעולין מן ג' פעמי' ז' כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |2||1||7 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ב||א||ז | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Next, take the 1, which is not divisible by 7, and write it above the 7 resulting from 31 times 7; it is 8 and its diagram is as follows: | ||
| + | |style="text-align:right;"|ואז קח הא' שלא חלקת לז' ושים עתה על הז' שעולה השת' מן א"ג פעם ז' <s>ויהיה</s> ויהיה ח' וצורתו כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |2||1||8 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ב||א||ח | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::We find that when I take 218 and divide it by 7, the result is 31 and 1 remains. | ||
| + | |style="text-align:right;"|נמצא כשלקחתי הח'א'ב' וחלקתי ממנ' כמה פעמי' ז' ועולה א"ג ונשאר א‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Then, I take 31 and multiply 7 times 31; the result is 217. | ||
| + | |style="text-align:right;"|ואז לקחתי הא"ג וזרקתי לתור הז' וחשבתי כמה <s>פ'</s> ז' פעמים א"ג ועולה ממנ' זא"ב | ||
| + | |- | ||
| + | | | ||
| + | ::Next, I take the remaining 1 and add it to the 7; the result is 8. | ||
| + | |style="text-align:right;"|ואז לקחתי הא' שנשאר כבר ו{{#annot:term|178,2383|zhZv}}זרקתי{{#annotend:zhZv}} על זה הז' והוה ח' <s>כמו</s> | ||
| + | |- | ||
| + | | | ||
| + | ::We find 218 as at the beginning. | ||
| + | |style="text-align:right;"|נמצא ח'א'ב' כמו בראשון | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | ::<span style=color:Green>[Illustration of the procedure:]</span> | ||
| + | ::{| | ||
| + | |- | ||
| + | |<span style="color:purple">3</span>1||rowspan="2"|<math>\scriptstyle\xrightarrow{{\color{Purple}{3\sdot7}}={\color{blue}{21}}}</math>||<span style="color:#0000FF>21</span><span style="color:purple">1</span>||rowspan="2"|<math>\scriptstyle\xrightarrow{{\color{Purple}{1\sdot7}}={\color{blue}{7}}}</math>||21<span style="color:#0000FF>7</span>||rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{Purple}{7+1}}={\color{blue}{8}}}</math>||21<span style="color:#0000FF>8</span> | ||
| + | |- | ||
| + | |<span style="color:purple">7</span> ||  <span style="color:purple">7</span>|| || | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | == Chapter Seven: Proportions == | ||
| + | |||
| + | |style="text-align:right;"|‫<ref>114v continue</ref><big>שער עירוכין</big> | ||
| + | |- | ||
| + | |This respectable chapter is called the chapter on proportion, which according to the general opinion is needed in order to count, to calculate, to make wise, to educate, to know and understand a thing from a thing, to evaluate a thing after thing, and to comprehend its foundation and its nature. | ||
| + | |style="width:45%; text-align:right;"|שער הנכבד ונקרא שער הערך אשר לכולי עלמא צריך למנות ולחשוב ולחכם ולהשכיל לדעת ולהבין דבר מתוך דבר ולהעריך דבר אחר דבר ולעמוד על יסודו ועל בוריו | ||
| + | |- | ||
| + | |If you buy a large amount of goods for a high price and you need to estimate a small amount of goods according to this price, meaning how much should cost the small amount of goods you bought according to this price? | ||
| + | |style="text-align:right;"|כיצד אם לקחת מקח גדול בדמים מרובים ואת צריך להעריך מקח קטן לפי אותה דמים פי' כמה מגיע לפי אותה הדמים למקח הקטן שקנית | ||
| + | |- | ||
| + | |Or vice versa, you buy a small amount of goods for a low price and you want to estimate a large amount of goods accordingly. | ||
| + | |style="text-align:right;"|או להיפך שקנית מקח קטן בדמים מועטי' ואתה רוצה להעריך אחריו מקח גדול | ||
| + | |- | ||
| + | |Here is how you calculate, count, and evaluate: | ||
| + | |style="text-align:right;"|הילך היאך תחשוב ותמנה ותעריך | ||
| + | |- | ||
| + | |Write the large amount of goods and write the price of the large amount of goods beneath the large amount of goods. Then, write the small amount of goods beneath the price. | ||
| + | |style="text-align:right;"|תכתו' המקח גדול וסכו דמים מן הגדול המקח תכתוב תחת המקח גדול ואח"כ תכתוב המקח הקטן תחת הסכום | ||
| + | |- | ||
| + | |Multiply the price of the large amount of goods you wrote above, below the large amount of goods, by the small amount of goods. Take the product and divide it, according to the chapter of division, by the large amount of goods; what you receive is the price of the small amount of goods. | ||
| + | |style="text-align:right;"|ותכפול דמי המקח הגדול אשר כתבת למעלה אחר המקח הגדול תכפול אותו על מניין מקח קטן והעולה קח בידך ותחלוק אותו בשער החילוק על המקח הגדול ומה שיעלה בידך כל כך ערך המקח הקטן | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | <math>\scriptstyle{\color{OliveGreen}{\frac{\left(price\ of\ large\ amount\ of\ goods\right)\times\left(small\ amount\ of\ goods\right)}{large\ amount\ of\ goods}}}</math> | ||
| + | |- | ||
| + | |If you are left with fractions that are less than the divisor, say: I have fractions left of which a certain amount is one unit of the required. | ||
| + | |style="text-align:right;"|ואם יותר לך חלקים שלא יעלו לחלק המבוקש אז תאמר עוד נשאר לי חלקים כך וכך שסכום כך וכך עולה לחלק אחד של המבוקש | ||
| + | |- | ||
| + | |Or vice versa, you want to evaluate [the price of] a large amount of goods according to [the price of] a smaller amount of the goods: write the small amount of goods above, then write the known price of this amount of goods and write the large amount of goods below, beneath the price of the small amount of goods. | ||
| + | |style="text-align:right;"|ואם להיפך שאתה רוצה להעריך חשבון מקח גדול אחר חשבון מקח קטן אז תכתוב חשבון הקטן למעלה ואח"כ תכתוב דמים הידועים לאותו מקח והחשבון הגדול תכתוב למטה <s>נ</s> תחת דמיו של חשבון הקטן | ||
| + | |- | ||
| + | |Multiply the large amount of goods by the known price of the small amount of goods, and divide the product, according to the chapter of division, by the small amount of goods above as above. | ||
| + | |style="text-align:right;"|ותכפול חשבון <s>הקטן</s> הגדול על דמים הידועי' של מקח הקטן והעולה תחלק בשער החילוק על מקח הקטן ‫<ref>115r</ref>שלמעלה כדלעיל | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | <math>\scriptstyle{\color{OliveGreen}{\frac{\left(price\ of\ small\ amount\ of\ goods\right)\times\left(large\ amount\ of\ goods\right)}{small\ amount\ of\ goods}}}</math> | ||
| + | |- | ||
| + | |This is rule [of the calculation procedure]: | ||
| + | |style="text-align:right;"|וזה הכלל | ||
| + | |- | ||
| + | |First, write the amount of goods you know. Then, write its price, and after it the amount of goods, [whose price] you wish to know and evaluate. | ||
| + | |style="text-align:right;"|המקח אשר ידעת תכתוב תחילה ואח"כ תכתוב דמיו ואח"כ המקח אשר בקשת לידע להעריך | ||
| + | |- | ||
| + | |Multiply, then divide as I have instructed you. | ||
| + | |style="text-align:right;"|ותכפול ואח"כ תעריך כמו שציויתיך | ||
| + | |- | ||
| + | | | ||
| + | === <span style=color:Green>Word Problems</span> === | ||
| + | | | ||
| + | |- | ||
| + | |Now I will write for you two or three examples, so you may understand and become wise. | ||
| + | |style="text-align:right;"|ועתה אכתוב לך ב' או ג' דמיונות כדי שתוכל להבין ולהשכיל | ||
| + | |- | ||
| + | ! | ||
| + | ==== <span style=color:Green>Pricing Problems - Find the Price</span> ==== | ||
| + | |||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:cloth|629|k21g}}A man who asks you: I bought 70 cubits of cloth for 40 dinar, how much will 55 cubits cost? | ||
| + | :<math>\scriptstyle\frac{70}{40}=\frac{55}{X}</math> | ||
| + | |style="text-align:right;"|איש אשר ישאלך הנה קניתי ע' אמות בגד עבור מ' דינרים כמה מגיע לנ"ה אמות{{#annotend:k21g}} | ||
| + | |- | ||
| + | | | ||
| + | ::First, write 70 cubits, then the price of the bargain, and then the 55 cubits, whose price you wish to know, as this: | ||
| + | |style="text-align:right;"|אז תכתוב תחילה הע' אמות ואח"כ דמי הע' אמות ואח"כ הנ"ה אמות אשר בקשת לידע ערכיהן כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |70 | ||
| + | |- | ||
| + | |40 | ||
| + | |- | ||
| + | |55 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |‫0ז | ||
| + | |- | ||
| + | |‫0ד | ||
| + | |- | ||
| + | |הה | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::<span style=color:Green>'''Rule of Three:'''</span> Multiply 55 by 40 and the result is 2200, as this: | ||
| + | |style="text-align:right;"|ותכפול נ"ה על מ' פי' תמנה ותחשוב כמה עולה נ"ה פעמי' מ' והעולה הוא ב' אלפי' וב' מאות כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |2||2||0||0 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ב||ב||‫0||‫0 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Then, divide 2200 by 70, meaning, divide according to the chapter of division: how many times 70 is in it? The division is 31 times 70 and 30 smaller parts of which 70 are a whole unit, i.e. a dinar. | ||
| + | |style="text-align:right;"|ואח"כ תחלק ב' אלפי' לע'ע' פי' חלוק בשער החילוק כמה פעמי' ע' יש בהן והחילוק הוא ל"א פעמי' ע' ול' חלקי' קטני' שע' הם חלק אחד דהיינו דינר | ||
| + | |- | ||
| + | | | ||
| + | ::We find the question that you were asked: when 70 cubits were bought for 40 dinar, how much will 55 cubits cost? [is solved by:] 31 dinar and 30 parts of which 70 are one dinar. | ||
| + | ::<math>\scriptstyle{\color{blue}{X=\frac{55\sdot40}{70}=\frac{2200}{70}=31+\frac{30}{70}}}</math> | ||
| + | |style="text-align:right;"|נמצא השאלה ששואל לך כשקוני' ע' אמו' עבור מ' דינרי' כמה מגיע לנ"ה ע' אמות השב לו מגיע לו ל"א דינרי' ועוד ל' חלקי' שע' מהם עושה חלק שלם דהוא דינר | ||
| + | |- | ||
| + | | | ||
| + | ::<span style=color:Green>'''Check:'''</span> If you think: where it is proven and where we are told it is so? Lest the ratio is not as you had calculated. | ||
| + | |style="text-align:right;"|ואם תעלה על דעתך מאן מוכח ומאן לימא לן דכך הוא שמא הערך אינו כמו שחשבת | ||
| + | |- | ||
| + | | | ||
| + | ::Think about the 15 cubits that exceed the 55, for from 55 to 70 there are 15. | ||
| + | ::<math>\scriptstyle{\color{blue}{70-55=15}}</math> | ||
| + | |style="text-align:right;"|אז צא וחשוב הט"ו אמות העודפי' על הנ"ה כי מנ"ה עד ע' יש ט"ו | ||
| + | |- | ||
| + | | | ||
| + | ::Evaluate: how much will 15 cubits cost, when 70 cubits are bought for 40 dinar? | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{70}{40}=\frac{15}{y}}}</math> | ||
| + | |style="text-align:right;"|ותעריך כמה מגיע לט"ו אמות כשע' באות עבור מ' דינרי‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Here is how you do it: | ||
| + | |style="text-align:right;"|והילך היאך <s>תע</s> תעשה | ||
| + | |- | ||
| + | | | ||
| + | ::Write 70 cubits, then 15 cubits, as this: | ||
| + | |style="text-align:right;"|תכתוב הע' אמות למעלה ואח"כ למטה הימנו המ' דינרי' ואח"כ הט"ו אמו' כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color: blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |70 | ||
| + | |- | ||
| + | |40 | ||
| + | |- | ||
| + | |15 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |‫0ז | ||
| + | |- | ||
| + | |‫0ד | ||
| + | |- | ||
| + | |הא | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::<span style=color:Green>'''Rule of Three:'''</span> Multiply 15 by 40, meaning: think how much is 15 times 40? The result is 600 | ||
| + | |style="text-align:right;"|ותכפול הט"ו על המ' פי' חשוב כמה עולה ט"ו פעמי' מ' ועולה ו' מאות | ||
| + | |- | ||
| + | | | ||
| + | ::Divide 600 to 70, meaning: count how many times 70 is in 600? The result is eight times 70 and 40 parts remain, of which 70 are a whole unit, meaning one dinar. | ||
| + | |style="text-align:right;"|ואח"כ תחלוק ו' מאות לע' ע' פי' תמנה כמה פעמי' ע' ישנו בו' מאות ויעלה שמנה פעמי' ע' ועוד ישארו מ' חלקי' שע' מהן עושי' חלק שלם פי' דינר אחד | ||
| + | |- | ||
| + | | | ||
| + | ::We find that when you buy 70 cubits for 40 dinar, 15 cubits are cost 8 dinar and 40 parts of which 70 are one dinar. | ||
| + | ::<math>\scriptstyle{\color{blue}{y=\frac{15\sdot40}{70}=\frac{600}{70}=8+\frac{40}{70}}}</math> | ||
| + | |style="text-align:right;"|נמצא כשקנית ע' אמות עבור מ' דנרים מגיע לט"ו אמו' ח' א' דנרים ומ' חלקי' שע' מהם עושי' חלק שלם דינר | ||
| + | |- | ||
| + | | | ||
| + | ::Above we calculated that 55 cost 31 dinar and 30 parts of which 70 are one dinar. | ||
| + | |style="text-align:right;"|ולמעלה חשבנו שמגיע לנ"ה אמות ל"א דינרי' ול' חלקי' שע' מהן עושי' חלק שלם פי' דינר אחד | ||
| + | |- | ||
| + | | | ||
| + | ::Now sum the prices together: 31 dinar and 8 dinar are 39 dinar. | ||
| + | |style="text-align:right;"|עתה חבר הערך יחד ל"א דינרי' וח' דינרי' הרי ל"ט דינרים | ||
| + | |- | ||
| + | | | ||
| + | ::Sum the 40 parts that remain for the 15 cubits with the 30 parts that remain for the 55, they are 70, i.e. a whole dinar. | ||
| + | |style="text-align:right;"|ומ' חלקי' הנותרי' בט"ו ול' חלקי' הנותרי' בנ"ה צרפם יחד ויהיו ע' דהיינו דינר שלם | ||
| + | |- | ||
| + | | | ||
| + | ::Add this dinar to the 39 and they are 40. | ||
| + | |style="text-align:right;"|תן אותו דינר עם הל"ט ויהיו ארבעי‫' | ||
| + | |- | ||
| + | | | ||
| + | ::We find that 55 cubits and 15 cubits cost 40 dinar. | ||
| + | |style="text-align:right;"|נמצא שנ"ה אמות וט"ו אמות באות עבור מ' דינרי‫' | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(31\frac{30}{70}\right)+\left(8+\frac{40}{70}\right)=\left(31+8\right)+\left(\frac{30}{70}+\frac{40}{70}\right)=39+\frac{70}{70}=39+1=40}}</math> | ||
| + | |- | ||
| + | | | ||
| + | ::Sum 55 and 15, it is 70. | ||
| + | ::<math>\scriptstyle{\color{blue}{55+15=70}}</math> | ||
| + | |style="text-align:right;"|צרוף נ"ה וט"ו ויהיה ע‫' | ||
| + | |- | ||
| + | | | ||
| + | :We find that now you know and understand that you calculated rightly and correctly. | ||
| + | |style="text-align:right;"|נמצא שעכשיו אתה יודע ומבין שחשבת כדין וכשורה וד"ל | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:barrels|629|xb4q}}Another example: If a person asks you: I bought 100 barrels for 90 liṭra, how much will one barrel cost? | ||
| + | :<math>\scriptstyle\frac{100}{90}=\frac{1}{X}</math> | ||
| + | |style="text-align:right;"|‫<ref>115v</ref>ערך אחר <big>אם</big> ישאלך אדם הנה קניתי ק' חבית בעד צ' ליטרין כמה מגיע לחבית אחת{{#annotend:xb4q}} | ||
| + | |- | ||
| + | | | ||
| + | ::First, write 100 barrels, then their price, i.e. 90 liṭra and beneath the 90 liṭra write one barrel, like this: | ||
| + | |style="text-align:right;"|אז תכתוב תחילה הק' חבית ואח"כ דמיהן דהיינו הצ' ליטרין ותחת הצ' ליטרין תכתוב החבית האחד כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color: blue; text-align:right;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |100 | ||
| + | |- | ||
| + | |90 | ||
| + | |- | ||
| + | |1 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:right;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |‫00א | ||
| + | |- | ||
| + | |‫0ט | ||
| + | |- | ||
| + | |א | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::<span style=color:Green>'''Rule of Three:'''</span> Multiply the bottom 1 by 90 that is above it, 1 times 90, the result is 90. | ||
| + | |style="text-align:right;"|ותכפול א' התחתונה על הצ' שלמעלה הימנו א' פעמי' צ' יעלה תשעים | ||
| + | |- | ||
| + | | | ||
| + | ::Then, divide the 90 by 100, meaning: how many times 100 is in 90? You will not get even one time 100. On the contrary, you are missing ten and this is the price of one barrel: 90 parts of which 100 are one liṭra. | ||
| + | ::<math>\scriptstyle{\color{blue}{X=\frac{1\sdot90}{100}=\frac{90}{100}}}</math> | ||
| + | |style="text-align:right;"|ואח"כ תחלק אותה צ' לק"ק פי' מנה כמה פעמי' ק' יש בצ' ולא תשיג ידך אפי' פעם אחת ק' אדרבה חסר לך י' וזה הערך המגיע לחבית א' צ' חלקי' שק' מהן עולין לטרא | ||
| + | |- | ||
| + | | | ||
| + | ::This our teacher R. Wiesel found in another book, in these words. | ||
| + | |style="text-align:right;"|זה מצא מהר"ר וייזיל בספר אחר וכך לשונו | ||
| + | |- | ||
| + | | | ||
| + | ::In my opinion there is no need for this premise, as a person does not think of dividing 90 parts of which 100 are a whole unit. | ||
| + | |style="text-align:right;"|ולפי דעתי שאין צריך לטעם זה כי אין מחשבתו של אדם לחילוק צ' חלקי' שק' מהן חלק אחד | ||
| + | |- | ||
| + | | | ||
| + | ::The premise that is more appropriate, in my opinion, in the Gentile calculations also, is as I will write below concerning the question "100 barrels for 90 liṭra, how much will one [barrel] cost?" | ||
| + | |style="text-align:right;"|וזה הטעם ראוי יותר בעיני גם חשבונ' של גוים נמי כזה שאכתוב הנה שאלת ק' חבית בעד ק' חבית בעד צ' ליטרי' כמה מגיע לאחד | ||
| + | |- | ||
| + | | | ||
| + | ::Write like this: | ||
| + | |style="text-align:right;"|כתוב כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color: blue; text-align:right;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |100 | ||
| + | |- | ||
| + | |90 | ||
| + | |- | ||
| + | |1 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:right;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |‫00א | ||
| + | |- | ||
| + | |‫0ט | ||
| + | |- | ||
| + | |א | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Think in your mind: how many pešuṭim there are in 90 liṭra, if in one liṭra there are 20 pešuṭim? | ||
| + | |style="text-align:right;"|חשוב בדעתך כמה פשי' יש בצ' ליטרי' אם הין אחד כ' פשי‫' | ||
| + | |- | ||
| + | | | ||
| + | ::<span style=color:Green>'''Rule of Three:'''</span> Multiply 20 times 90, the result is 1800, as this: | ||
| + | |style="text-align:right;"|חשוב ב' פעמי' ב' ויעלה ח' מאות ואלף כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |1||8||0||0 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |א||ח||‫0||‫0 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Think according to the chapter of division: how many times 100 is in 1800? You will find 18 times exactly and so is the price: 18 pešuṭim for one barrel. | ||
| + | ::<math>\scriptstyle{\color{blue}{X=\frac{1\sdot\left(90\sdot20\right)}{100}=\frac{1800}{100}=18}}</math> | ||
| + | |style="text-align:right;"|חשוב בשער החילוק כמה פעמי' מאה יש בח' מאות ואלף ותמצא י"ח פעמי' מכוון כך היא החשבון י"ח פשי' לאחד | ||
| + | |- | ||
| + | | | ||
| + | :And if you have small parts remaining, say: such and such parts, of which so and so are a whole unit, meaning one pašuṭ. Note well. | ||
| + | |style="text-align:right;"|ואם ישארו לך חלקי' בקטני' אמור כך וכך חלקי' שכך וכך עלו לחלק שלם פי' לטרא ודו"ק | ||
| + | |- | ||
| + | |We wish to evaluate: | ||
| + | |style="text-align:right;"|ערך אחר <big>הנה</big> בקשנו להעריך | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:cloth|629|3eLi}}If a person asks: I bought 19 cubits of cloth for 13 dinar, how much will 13 cubits cost? | ||
| + | :<math>\scriptstyle\frac{19}{13}=\frac{13}{X}</math> | ||
| + | |style="text-align:right;"|אם ישאל אדם קניתי י"ט אמות בגד עבור י"ג דינרי' כמה מגיע לי"ג אמות{{#annotend:3eLi}} | ||
| + | |- | ||
| + | | | ||
| + | ::Evaluate as follows: | ||
| + | |style="text-align:right;"|אז תעריך כך | ||
| + | |- | ||
| + | | | ||
| + | ::First, write 19 cubits, then their price, i.e. 13 dinar, then 13 cubits, like this: | ||
| + | |style="text-align:right;"|כתוב תחילה י"ט אמות ואח"כ דמיו דהיינו י"ג דינרי' ואח"כ י"ג אמות כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color: blue; text-align:right;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |19 | ||
| + | |- | ||
| + | |13 | ||
| + | |- | ||
| + | |13 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:right;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |טא | ||
| + | |- | ||
| + | |גא | ||
| + | |- | ||
| + | |גא | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::<span style=color:Green>'''Rule of Three:'''</span> Multiply 13 above by 13 below, meaning: calculate how much is 13 times 13, you will find that the result is 169, as this: | ||
| + | |style="text-align:right;"|ותכפול י"ג של מעלה על י"ג שלמטה פי' מנה כמה עולה י"ג פעמי' ותמצא שיעלה קס"ט כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |1||6||9 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |א||ו||ט | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Then, divide 169 by 19, meaning: how many times 100 is in 1800? You will find that there are 8 times 19 in it and 17 parts remain, of which 19 are a whole unit. | ||
| + | |style="text-align:right;"|ואז תחלק קס"ט לי"ט י"ט פי' מנה כמה פעמי' י"ט בקס"ט ותמצא שיש בו שיש בו ח' פעמי' י"ט ונשארו י"ז חלקי' שי"ט מהן עולה חלק אחד | ||
| + | |- | ||
| + | | | ||
| + | ::This is the price of 13 cubits: when 19 cubits are bought for 13 dinar, 13 cubits cost 8 dinar and 17 parts, of which 19 are a whole unit, i.e. one dinar. | ||
| + | ::<math>\scriptstyle{\color{blue}{X=\frac{13\sdot13}{19}=\frac{169}{19}=8+\frac{17}{19}}}</math> | ||
| + | |style="text-align:right;"|וזה הערך המגיע לי"ג אמות כשקוני' י"ט אמות עבור י"ג דינרי' ח' דנרים וי"ז חלקי' קטני' שי"ז מהן עולין חלק אחד דהיינו דינר אחד | ||
| + | |- | ||
| + | |Likewise, if you wish to evaluate a large amount of merchandise through a small amount of merchandise, I will teach how: | ||
| + | |style="text-align:right;"|<big>ו</big>כן אם תרצה להעריך מקח גדול אחד ממקח קטן אשכילך היאך תעשה | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:cubits|629|xlAl}}I bought 21 cubits of cloth for 43 dinar, how much will 65 cubits cost? | ||
| + | :<math>\scriptstyle\frac{21}{43}=\frac{65}{X}</math> | ||
| + | |style="text-align:right;"|אם ישאל השואל הנה קניתי כ"א אמות בגד עבור מ"ג דינרי' כמה מגיע לס"ה אמות{{#annotend:xlAl}} | ||
| + | |- | ||
| + | | | ||
| + | ::First, write 21 cubits, then 43 dinar, then 65 cubits that you wish to evaluate, like this: | ||
| + | |style="text-align:right;"|כתוב בתחילה הכ"א אמות ואח"כ המ"ג דינרי' ואח"כ הס"ה אמות ‫<ref>116r</ref>אשר בקשת לחשוב כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color: blue; text-align:left;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |small amount of merchandise||21 | ||
| + | |- | ||
| + | |price||43 | ||
| + | |- | ||
| + | |large amount of merchandise||65 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:right;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |מקח קטן||אב | ||
| + | |- | ||
| + | |מעות||גד | ||
| + | |- | ||
| + | |מקח גדול||הו | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::<span style=color:Green>'''Rule of Three:'''</span> Multiply 65 by 43, meaning how much is 43 times 65, the result is 2795. | ||
| + | |style="text-align:right;"|ותכפול ה"ו על ג"ד פי' כמה עולה ג"ד פעמי' ה"ו ויעלה <s>הטז"ב</s> הטז"ב | ||
| + | |- | ||
| + | | | ||
| + | ::Then, divide 2795 according to the chapter of division: how many times 21 is [in it]? You find that there are 133 times 21 in it and 2 parts remain, of which 21 are a whole unit, i.e. one dinar. | ||
| + | |style="text-align:right;"|ואח"כ חלוק הטז"ב בשער החילוק כמה פעמי' שיש בהן א"ב ותמצא ג'ג'א' פעמי' ועוד נשארו ב' חלקי' קטני' שא"ב מהן עושי' חלק אחד דהיינו דינר | ||
| + | |- | ||
| + | | | ||
| + | ::We find that if you bought 21 cubits for 43 dinar, 65 cubits cost 133 dinar and 2 parts, of which 21 are one dinar. | ||
| + | ::<math>\scriptstyle{\color{blue}{X=\frac{65\sdot43}{21}=\frac{2795}{21}=133+\frac{2}{21}}}</math> | ||
| + | |style="text-align:right;"|נמצא אם קנית א"ב אמות עבור ג"ד דנרי' מגיע לה"ו אמות ג'ג'א' דינרי' וב' חלקי' שא"ב מהן דינר | ||
| + | |- | ||
| + | | | ||
| + | ::<span style=color:Green>'''Check:'''</span> If you wish to know if you calculated correctly or not. | ||
| + | |style="text-align:right;"|ואם תרצה לידע אם אמת חשבת אם לאו | ||
| + | |- | ||
| + | | | ||
| + | ::See by how much 65 exceeds 21, i.e. by 44. | ||
| + | ::<math>\scriptstyle{\color{blue}{65-21=44}}</math> | ||
| + | |style="text-align:right;"|הבט וראה כמה ה"ו עולה יותר מא"ב דהיינו ד"ד | ||
| + | |- | ||
| + | | | ||
| + | ::<span style=color:Green>'''Rule of Three:'''</span> [Multiply] 44 by 43, according to the chapter of division, meaning: 43 times 44, i.e. 1892. | ||
| + | |style="text-align:right;"|השליכהו בשער הכפל על ג"ד פי' ג"ד פעמי' ד"ד דהיינו בטח"א | ||
| + | |- | ||
| + | | | ||
| + | ::Then, divide it, according to the chapter of division: how many times 21 is in it? We find 90 times and 2 small parts remain, of which 21 is a whole unit. | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{44\sdot43}{21}=\frac{1892}{21}=90+\frac{2}{21}}}</math> | ||
| + | |style="text-align:right;"|ואח"כ חלקיהו בשער החילוק כמה פעמי' יש בהן א"ב ותמצא 0"ט פעמי' ועוד נשארו ב' חלקי' קטני' שא"ב מהן חלק אחד שלם | ||
| + | |- | ||
| + | | | ||
| + | ::Add 90 and 2 small parts to 43, i.e. the dinar for the 21 cubits, and it is 133 and 2 small parts, like this: | ||
| + | ::<math>\scriptstyle{\color{blue}{43+\left(90+\frac{2}{21}\right)=133+\frac{2}{21}}}</math> | ||
| + | |style="text-align:right;"|חבר 0"ט וב' חלקי' קטני' על ג"ד דהיינו הדינרי' השייכי' לא"ב זהו ויהיה גג"א וב' חלקי' קטני' כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color: blue; text-align:right;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |133 | ||
| + | |- | ||
| + | |2 | ||
| + | |- | ||
| + | |43 | ||
| + | |- | ||
| + | |90 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:right;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |גגא | ||
| + | |- | ||
| + | |ב | ||
| + | |- | ||
| + | |גד | ||
| + | |- | ||
| + | |‫0ט | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | :So, the calculation is correct. Note well. | ||
| + | |style="text-align:right;"|והיינו כוונת החשבון ולמבין די | ||
| + | |- | ||
| + | ! | ||
| + | |||
| + | ==== <span style=color:Green>Boiling Problem</span> ==== | ||
| + | |||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :A question that is derived from this chapter on proportion: | ||
| + | |style="text-align:right;"|שאלה היוצא משער הערך הזה | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:WP|663|VxeG}}You have 50 se'ah [1 se'ah = ca. 22 liter], and you say: when I boil them on fire, while boiling 7 se'ah evaporate each day, if 5 se'ah overflowed, and we reduce the heat accordingly, how much will be evaporated from the remaining 45 se'ah each day? | ||
| + | :<math>\scriptstyle\frac{50}{7}=\frac{50-5}{X}</math> | ||
| + | :<math>\scriptstyle\frac{50}{7}=\frac{45}{X}</math> | ||
| + | |style="text-align:right;"|הנה לפניך נ' סאין מים ואם אתה ש' אבשל אות' על האור מתבשלין ונחסרי' בכל יום ז' סאין ועתה שואלי' <s>ועתה</s> אם נשכפו ה' סאין ואנו ממעטי' האור לפי זה כמה יחסרו המ"ה סאין הנותרי' ביום אחד{{#annotend:VxeG}} | ||
| + | |- | ||
| + | | | ||
| + | ::Here is how you do it: | ||
| + | |style="text-align:right;"|הילך היאך תעשה | ||
| + | |- | ||
| + | | | ||
| + | ::First, write 50 se'ah, then write 7 se'ah that evaporate each day and beneath the 7 se'ah write the remaining 45 se'ah, like this: | ||
| + | |style="text-align:right;"|תכתוב תחילה הנ' סאין ואח"כ תכתוב הז' סאין הנחסרי' ביום אחד ותחת הז' סאין תכתוב המ"ה סאין הנשארי' כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color: blue; text-align:right;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |50 | ||
| + | |- | ||
| + | |7 | ||
| + | |- | ||
| + | |45 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:right;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |‫0ה | ||
| + | |- | ||
| + | |ז | ||
| + | |- | ||
| + | |הד | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::<span style=color:Green>'''Rule of Three:'''</span> Multiply what is beneath by 7 that is above it, meaning, calculate: how much is 45 times 7? The result is 315. | ||
| + | |style="text-align:right;"|ותכפול מ"ה שלמטה על ז' שלמעלה הימנה פי' חשוב כמה עולה מ"ה פעמי' ז' ויעלה שט"ו | ||
| + | |- | ||
| + | | | ||
| + | ::Divide it by 50, meaning, calculate: how many times 50 there are in 315? You find in it 6 times 50 and 15 parts remain, of which 50 is a whole unit. | ||
| + | ::<math>\scriptstyle{\color{blue}{X=\frac{45\sdot7}{50}=\frac{315}{50}=6+\frac{15}{50}}}</math> | ||
| + | |style="text-align:right;"|תחלוק אות' שט"ו לנ' פי' כמה פעמי' יש בשט"ו ותמצא בו ו' פעמי' נ' ועוד נשארו ט"ו חלקי' קטני' שנ' מהן חלק אחד שלם | ||
| + | |- | ||
| + | | | ||
| + | ::We find that when 50 se'ah are reduced by 7 se'ah each day, 45 se'ah are reduced by 6 se'ah and 15 parts, of which 50 are a whole se'ah. | ||
| + | |style="text-align:right;"|נמצא כשנ' סאין יחסרו ז' סאין ביום אחד מ"ה סאין יחסרו ו' סאין וט"ו חלקי' קטני' שנ' מהן סיאה שלם | ||
| + | |- | ||
| + | | | ||
| + | :Another question: | ||
| + | |style="text-align:right;"|שאלה אחרת | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:WP|663|8v4H}}If one asks: there are 91 se'ah of water, when boiling on fire 9 se'ah evaporate each day, if we add to them 11 se'ah more, so there are now 102 se'ah and we increase the heat accordingly - since, if we would not increase the heat, the water would not evaporate according to this calculation, as the heat is not dominant much when the water is plentiful as when the water is low, hence when adding water, one should increase the heat, and if the water is reduced, one should reduce the heat - back to the question asked: how much will be evaporated from the 102 se'ah each day? | ||
| + | :<math>\scriptstyle\frac{91}{9}=\frac{91+11}{X}</math> | ||
| + | :<math>\scriptstyle\frac{91}{9}=\frac{102}{X}</math> | ||
| + | |style="text-align:right;"|<big>אם</big> ישאל השואל הנה יש צ"א סאין מים ואם מתבשלין על האור יחסרו ביום אחד ט' סאין ואם נוסיף עליהם עוד י"א סאין שיהיה הכל ק"ב סאי' ואנו מרבין את האש לפי זה שאם לא היינו מרבי' את האש אז לא היו המים מתמעטי' וחסירי' לפי החשבון הזה כי האור אינו שולט כל כך כשהמים הרבה כמו שאם היו המים מועטי' לפיכך כשמרבי' המים אז ‫<ref>116v</ref>צריכי' להרבות האש או אם מתמעטי' אז צריכי' למעט האש<br> | ||
| + | נחזור לשאלה ששאלנו כמה יחסרו ק"ב סאין ביו' אחד כשצ"א חסירי' ט' סאין{{#annotend:8v4H}} | ||
| + | |- | ||
| + | | | ||
| + | ::Here is how you do it: | ||
| + | |style="text-align:right;"|הילך היאך תעשה | ||
| + | |- | ||
| + | | | ||
| + | ::First, write 91 se'ah, then write 9 se'ah, then 102 beneath, like this: | ||
| + | |style="text-align:right;"|תכתוב תחילה הצ"א ואח"כ הט' ואח"כ הק"ב כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color: blue; text-align:right;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |91 | ||
| + | |- | ||
| + | |9 | ||
| + | |- | ||
| + | |102 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:right;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |אט | ||
| + | |- | ||
| + | |ט | ||
| + | |- | ||
| + | |ב0א | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::<span style=color:Green>'''Rule of Three:'''</span> Multiply 102 beneath by 9 above, meaning: how much is 9 times 102? The result is 918. | ||
| + | |style="text-align:right;"|ותכפול ק"ב שלמט' על ט' שלמעלה פי' כמה עולה ט' פעמי' ק"ב ויעלה תתקי"ח | ||
| + | |- | ||
| + | | | ||
| + | ::Divide 918 by 91, meaning: how many times 91 there are in 918? There are 10 times 91 and 8 small parts, of which 91 is a whole unit. | ||
| + | ::<math>\scriptstyle{\color{blue}{X=\frac{102\sdot9}{91}=\frac{918}{91}=10+\frac{8}{91}}}</math> | ||
| + | |style="text-align:right;"|תחלוק תתקי"ח לצ"א פי' כמה פעמי' צ"א יש בתתקי"ח והיינו י' פעמי' צ"א וח' חלקי' קטנים שצ"א מהן חלק שלם | ||
| + | |- | ||
| + | | | ||
| + | ::We find that when 91 [se'ah] are reduced by 9 se'ah each day, 102 [se'ah] are reduced by 10 se'ah and 8 parts, of which 91 are a whole [unit]. | ||
| + | |style="text-align:right;"|נמצא כשצ"א חסירי' ביום אחד ט' סאין יחסרו ק"ב י' סאין וח' חלקי' שצ"א מהן עולי' חקל שלם | ||
| + | |- | ||
| + | |The checking procedure is as written above regarding the cloth. | ||
| + | |style="text-align:right;"|והמאזנים על זה כמו שכתבתי לעיל עם הבג‫' | ||
| + | |- | ||
| + | |This is the rule: the [boiling] water problems are similar to the cloth [pricing] problems, therefore their checking procedure and solving method are similar. | ||
| + | |style="text-align:right;"|זה הכלל זה השאלות עם המים אות באות כמו השאלו' עם הבגד לכן יש להם מאזני צדק אחד וחשבון אחד ול"ד | ||
| + | |- | ||
| + | ! | ||
| + | |||
| + | ==== <span style=color:Green>Find a Quantity Problems - Whole from Parts</span> ==== | ||
| + | | | ||
| + | |- | ||
| + | |Many sciences and problems are derived from this chapter that cannot be counted. | ||
| + | |style="text-align:right;"|והרבה חכמות ושאלות יוצאין משער זה אשר לא יוכל הסופר לכתוב | ||
| + | |- | ||
| + | |Now, I will explain a few of them with God's help. | ||
| + | |style="text-align:right;"|ועתה אפרש קצת ממנה בע"ה | ||
| + | |- | ||
| + | |I start by saying: | ||
| + | |style="text-align:right;"|‫[ובזה אתחיל ואומר]‫<ref>M om.</ref> | ||
| + | |- | ||
| + | !<span style=color:Green>Tree</span> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:tree|650|d8Xb}}1) You have a tree - a half of it is [ingrained] in the ground, a third of it is in the water, and 5 cubits of it are up above the water, how much is the length of the whole tree? | ||
| + | :<math>\scriptstyle\frac{1}{2}X+\frac{1}{3}X+5=X</math> | ||
| + | |style="text-align:right;"|<big>א הנה</big> לך אילן שחציו בארץ ושלישיתו במים ומעל המים הוא ה' אמות<br> | ||
| + | כמה ארכו של כל האילן{{#annotend:d8Xb}} | ||
| + | |- | ||
| + | | | ||
| + | ::Here is how you do and understand the matter: | ||
| + | |style="text-align:right;"|הילך היאך תעשה ותבין העניין | ||
| + | |- | ||
| + | | | ||
| + | ::<span style=color:Green>'''Common denominator:'''</span> think, which is the smallest number that is divisible into 2 parts and 3 parts? It is 6. | ||
| + | |style="text-align:right;"|חשוב כל כך איזה חשבון הקרוב שיכולין לחלק לשני חלקי' ולג' חלקי' זה ו‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Subtract a half from 6; 3 remains. Subtract also a third; 1 remains. | ||
| + | ::<math>\scriptstyle{\color{blue}{6-\left(\frac{1}{2}\sdot6\right)-\left(\frac{1}{3}\sdot6\right)=3-\left(\frac{1}{3}\sdot6\right)=1}}</math> | ||
| + | |style="text-align:right;"|הסר מן ו' החציה וישאר ג' הסר ג"כ השלישי וישאר א‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Write the 1 first; after it, to the left, write 6; and beneath the 1 write 5 cubits, like this: | ||
| + | |style="text-align:right;"|אותה א' תכתוב תחילה ואחריה בצד שמאלית אצל הא' תכתוב ו' ותחת הא' תכתוב ה' אמות כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color: blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |6||1 | ||
| + | |- | ||
| + | | ||5 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ו||א | ||
| + | |- | ||
| + | | ||ה | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::<span style=color:Green>'''Rule of Three:'''</span> Multiply the 5 beneath the 1 by 6 that is next to the 1 on the top row, meaning: count how much is 5 times 6. The result is 30. | ||
| + | |style="text-align:right;"|ותכפול ה' שתחת הא' על ו' שעומד אחר הא' בשיטה עליונה פי' מנה כמה עולה ה' פעמי' ו' והעולה הוא ל‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Divide the 30 by 1, meaning: count how many times 1 there are in 30. It is a thing known to all that there are 30 times 1 in 30. Thus, this is the length of the tree, about which you ask: if [its half] is [ingrained] in the ground, its third is in the water, and 5 cubits [of it] are up above the water, and you ask how much is its length? Its length is 30 cubits, as this diagram: | ||
| + | ::<math>\scriptstyle{\color{blue}{X=\frac{5\sdot6}{1}=\frac{30}{1}=30}}</math> | ||
| + | |style="text-align:right;"|אות' ל' תחלק לא'א' פי' מנה כמה פעמי' א' יש בל' ודבר ידוע הוא לכל שיש בל' ל' פעמי' א' וזהו אורך האילן אשר שאלת אם תצא בארץ ושלישו עומד במים ומעל המים הוא ה' אמות וששאלת כמה ארכו הנה ארכו ל' אמות והדמיון כך כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color: blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |3||0 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ג||‫0 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::<span style=color:Green>'''Check:'''</span> If you fear that is not so, take a lance, whose length is 30 cubits. | ||
| + | |style="text-align:right;"|ואם אתה ירא שאינו כך אז קח רומח שארכו ל' אמו‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Subtract its half from it; it is 15. | ||
| + | |style="text-align:right;"|הסר ממנו חציו זהו ט"ו | ||
| + | |- | ||
| + | | | ||
| + | ::Then, subtract a third from it; it is ten. | ||
| + | |style="text-align:right;"|אח"כ הסר ממנו השליש זהו עשרה | ||
| + | |- | ||
| + | | | ||
| + | ::[Ten] and 15 are 25. | ||
| + | |style="text-align:right;"|וט"ו הרי כ"ה | ||
| + | |- | ||
| + | | | ||
| + | ::We find that the remainder is but 5 cubits. | ||
| + | ::<math>\scriptstyle{\color{blue}{30-\left(\frac{1}{2}\sdot30\right)-\left(\frac{1}{3}\sdot30\right)=30-15-10=30-25=5}}</math> | ||
| + | |style="text-align:right;"|נמצא שאין נשאר כ"א ה' אמות | ||
| + | |- | ||
| + | |Another example, in order that the reader will be well taught in it and understand its virtues and profit, and it is the question asked: | ||
| + | |style="text-align:right;"|דומיון אחר למען ירוץ הקורא בו ויבין מעלתו וטובו והוא שאלה ששואלין | ||
| + | |- | ||
| + | !<span style=color:Green>Lance</span> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:lance|650|KMWF}}A lance is standing one third, a quarter, and one fifth [embedded] in the ground, and its length above the ground is 7 cubits, how much is the length of the whole lance? | ||
| + | :<math>\scriptstyle\frac{1}{3}X+\frac{1}{4}X+\frac{1}{5}X+7=X</math> | ||
| + | |style="text-align:right;"|הנה לך רומח שעומד השליש והרביעי' והחומש בארץ ולמעלה מן הארץ ארכו ז' אמות <br> | ||
| + | כמה ארכו של כל הרומח {{#annotend:KMWF}} | ||
| + | |- | ||
| + | | | ||
| + | ::<span style=color:Green>'''Common denominator:'''</span> think of a number that has a third, a quarter and a fifth; it is 60, which is divisible by 3, 4 and 5. | ||
| + | |style="text-align:right;"|אז חשוב איזה חשבון שיש בו <ref>117r</ref>שליש ורביע וחומש וזהו ס' שיכולי' לחלק לג' ולד' ולה‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Subtract from a third from 60; it is 20. | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{1}{3}\sdot60=20}}</math> | ||
| + | |style="text-align:right;"|והסר מס' שליש וזהו כ‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Subtract also a quarter; it is 15. | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{1}{4}\sdot60=15}}</math> | ||
| + | |style="text-align:right;"|הסר כמו כן הרביע הוא ט"ו | ||
| + | |- | ||
| + | | | ||
| + | ::Subtract also a fifth; it is 12. | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{1}{5}\sdot60=12}}</math> | ||
| + | |style="text-align:right;"|והסיר ג"כ החומש זהו י"ב | ||
| + | |- | ||
| + | | | ||
| + | ::The total is 47. | ||
| + | ::<math>\scriptstyle{\color{blue}{20+15+12=47}}</math> | ||
| + | |style="text-align:right;"|ס"ה מ"ז | ||
| + | |- | ||
| + | | | ||
| + | ::You are left with 13 from the 60. | ||
| + | ::<math>\scriptstyle{\color{blue}{60-47=13}}</math> | ||
| + | |style="text-align:right;"|עוד נשאר לך מס' י"ג | ||
| + | |- | ||
| + | | | ||
| + | ::First, write the remaining 13. After the 13, slightly far from it, write the 60 and beneath the 13 write 7 cubits of the lance above the ground, like this: | ||
| + | |style="text-align:right;"|אז תכתוב תחלה י"ג שנשארו ואחר הי"ג בצדו רחוק מעט תכתוב הס' ותחת הי"ג תכתוב ז' אמות שהרומח עומד למעלה מן הארץ ויהיה כך | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color: blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |60||13 | ||
| + | |- | ||
| + | | ||7 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |‫0ו||גא | ||
| + | |- | ||
| + | | ||ז | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::<span style=color:Green>'''Rule of Three:'''</span> Multiply 7 below by 60 on the top row, meaning: count how much is 7 times 60. The result is 420. | ||
| + | |style="text-align:right;"|תכפול ז' שלמטה על 0"ו שבשיטה עליונה פי' חשוב ומנה כמה עולה ז"פ 0"ו ויעלה ת"ך | ||
| + | |- | ||
| + | | | ||
| + | ::Divide the 420 by 13, meaning: count how many 13 there are in 420. You find that there are 32 times 13 in it and 4 remains that cannot become 13, which are 4 parts of 13 in a whole unit. | ||
| + | ::<math>\scriptstyle{\color{blue}{X=\frac{7\sdot60}{13}=\frac{420}{13}=32+\frac{4}{13}}}</math> | ||
| + | |style="text-align:right;"|תחלק ת"ך לי"ג י"ג פי' חשוב ומנה כמה י"ג יש בת"ך ותמצא שיש בו ל"ב פעמי' י"ג ועוד נשארו ד' שלא יכולנו לעשות מהם י"ג והם ד' מי"ג בשלם | ||
| + | |- | ||
| + | | | ||
| + | ::We find that the required lance, whose third, quarter, and fifth are [embedded] in the ground, and its [length] above the ground is 7 cubits, and you ask how much is the length of the whole lance? - its length is 32 cubits and 4 parts, of which 13 is a whole unit. | ||
| + | |style="text-align:right;"|נמצא שהרומח ששאלת כשהשליש והרביע והחומש בארץ ולמעלה מן הארץ אכרו ז' אמות ושאלת כמה אורך של כל הרומח ארכו ל"ב אמות וד' חלקי' שי"ג מהן חלק שלם | ||
| + | |- | ||
| + | !<span style=color:Green>Wall</span> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:wall|650|z5yG}}2) Another question: a wall whose base is buried 5 cubits in the ground, and it is a half, one third, and a seventh up above the ground, how much is the height of the whole wall? | ||
| + | :<math>\scriptstyle\frac{1}{2}X+\frac{1}{3}X+\frac{1}{7}X+5=X</math> | ||
| + | |style="text-align:right;"|<big>ב שאלה</big> אחרת הנה חומה שיסודה שקוע' בקרקע ה' אמו' ולמעלה מן הארץ היא גבוה החציה והשלישי והשביעי‫'<br> | ||
| + | כמה גובהה של כל החומה{{#annotend:z5yG}} | ||
| + | |- | ||
| + | | | ||
| + | ::<span style=color:Green>'''Common denominator:'''</span> think, which is the number that can be easily divided by 2, 3 and 7. | ||
| + | |style="text-align:right;"|אז חשוב איזה חשבון שיכולין לחלקו לב' ולג' ולז' בקל לפי רגע | ||
| + | |- | ||
| + | | | ||
| + | ::Here is a way how you can find this number that is divisible by 2, 3 and 7: count 7 times 3, the result is 21. | ||
| + | |style="text-align:right;"|והנה לך דרך היאך תמצא אותו חשבון המתחלק לב' ולג' ולז' תמנה זפ"ג כמה כ"א | ||
| + | |- | ||
| + | | | ||
| + | ::21 times two are 42. Thus, 42 is divisible by two, three and seven. | ||
| + | ::<math>\scriptstyle{\color{blue}{2\sdot3\sdot7=2\sdot21=42}}</math> | ||
| + | |style="text-align:right;"|כ"א פעמי' שנים כמה הם מ"ב הרי שמ"ב מתחלקי' לשנים ולשבעה ולשלשה | ||
| + | |- | ||
| + | | | ||
| + | ::Or, by this way and all is the same: 2 times 3 are 6; 6 times 6 are 36; and you already have a 6, so sum 6 with 36, it is 42. | ||
| + | ::<math>\scriptstyle{\color{blue}{2\sdot3\sdot7=\left(6\sdot6\right)+6=36+6=42}}</math> | ||
| + | |style="text-align:right;"|‫[או כלך לדרך זו והכל אחד ב' פעמים ג' הרי ו' ו' פעמים ו' הרי ל"ו וכבר היו לך ו' צרוף ו' עם ל"ו הרי מ"ב הוא‫] | ||
| + | |- | ||
| + | | | ||
| + | ::Or, by this way and all is the same: calculate 2 times 3; it is 6. 6 times 7 is 42. | ||
| + | ::<math>\scriptstyle{\color{blue}{2\sdot3\sdot7=6\sdot7=42}}</math> | ||
| + | |style="text-align:right;"|או נלך לדרך זו והכל אחד חשוב בפ"ג הרי ו' ו'פ"ז הרי מ"ב | ||
| + | |- | ||
| + | | | ||
| + | ::We find that 42 is divisible by seven, [three, and two]. | ||
| + | |style="text-align:right;"|נמצא שמ"ב הוא דבר המתחלק הוא לשביעיו' או לתשיעיות ולעשיריו‫' | ||
| + | |||
| + | |- | ||
| + | | | ||
| + | ::<span style=color:Green>'''Finding the least common multiple:'''</span> likewise, when you are asked for a thing that is divisible by seven, nine, ten, or any thing else that is divisible, always multiply this divisor by the other. | ||
| + | |style="text-align:right;"|‫[וכן לעולם כשישאלך דבר המתחלק לשביעי ולתשיעי ולעשירי‫] או לכל דבר המתחלק אז תכפול אותו חלק על האחד | ||
| + | |||
| + | |- | ||
| + | | | ||
| + | ::For instance, if one asks for a thing that is divisible by ten and nine: multiply 10 by 9, meaning: count how much is 10 times 9. | ||
| + | ::<math>\scriptstyle{\color{blue}{10\sdot9}}</math> | ||
| + | |style="text-align:right;"|כגון אם שאל דבר המתחלק לעשיריו' ולתשיעיו' אז תכפול י' על ט' פי' מנה כמה עולה י' פעמי'‫' | ||
| + | |- | ||
| + | | | ||
| + | ::If you are asked for a thing that is divisible by five and four, or hundreds, or thousands; or any thing that is divisible, multiply one by the other, or any thing that one wants it to be divisible by such and such, multiply one by the other. | ||
| + | |style="text-align:right;"|ואם ישאלך דבר המתחלק לחמישיות ולרביעיו' או למאות ולאלפי' או לכל דבר המתחלק תכפול זה על זה או לכל דבר שישאל השואל שמתחלק כך וכך תכפול זה על זה | ||
| + | |- | ||
| + | | | ||
| + | ::If one asks for a thing divisible by 3, 4, and 5: multiply 3 by 4, then multiply the result by 5; or vice versa, multiply 5 by 4 first, then multiply the result by 3. | ||
| + | ::<math>\scriptstyle{\color{blue}{3\sdot4\sdot5}}</math> | ||
| + | |style="text-align:right;"|ואם ישאל השואל דבר המתחלק לג' ד' וה' אז תכפול ג' על ד' והעולה שיעלה תכפול על ה' או איפכא תכפול תחילה ה' על ד' והעולה שיעלה בידך תכפול על ג‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Returning to our question above: the wall that is a half, one third, and a seventh up above the ground and its base is buried 5 cubits in the ground, how much is the height of the whole wall? | ||
| + | |style="text-align:right;"|ועתה נחזור לשאילתינו דלעיל החומה אשר היא חצייה ושליש ושביעי' גבוה על הקרקע ויסודה שקועה בקרקע ה' אמות כמה גובהה של כל החומה | ||
| + | |- | ||
| + | | | ||
| + | ::We find that we aske for a thing that is divisible by 2, 3 and 7: 2 times 3 is 6 and 6 times 7 is 42. We find that 42 is a number divisible by 2, 3 and 7. | ||
| + | ::<math>\scriptstyle{\color{blue}{2\sdot3\sdot7=6\sdot7=42}}</math> | ||
| + | |style="text-align:right;"|נמצא ששאלנו על דבר המתחלק ‫<ref>117v</ref>לב' ולג' ולז' ב"פ ג' היינו ו' ופ"ז היינו מ"ב<br> | ||
| + | נמצא שמ"ב הוא חשבון המתחלק לב' ולג' ולז‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Now, we subtract from 42 a half; it is 21. | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{1}{2}\sdot42=21}}</math> | ||
| + | |style="text-align:right;"|ועתה {{#annot:term|181,2384|jTqA}}נסיר{{#annotend:jTqA}} החציה ממ"ב והוא כ"א | ||
| + | |- | ||
| + | | | ||
| + | ::We subtract also a third from 42; it is 14. | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{1}{3}\sdot42=14}}</math> | ||
| + | |style="text-align:right;"|נסיר כמו כן השליש ממ"ב זהו יהיה <s>מ"ב</s> י"ד | ||
| + | |- | ||
| + | | | ||
| + | ::We subtract also a seventh from 42; it is 6. | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{1}{7}\sdot42=6}}</math> | ||
| + | |style="text-align:right;"|נסיר כמו כן השביעי' ממ"ב זהו יהיה ו‫' | ||
| + | |- | ||
| + | | | ||
| + | ::The total is 41. | ||
| + | ::<math>\scriptstyle{\color{blue}{21+14+6=41}}</math> | ||
| + | |style="text-align:right;"|ס"ה מ"א | ||
| + | |- | ||
| + | | | ||
| + | ::1 remains from the 42. | ||
| + | ::<math>\scriptstyle{\color{blue}{42-41=1}}</math> | ||
| + | |style="text-align:right;"|עוד נשאר ממ"ב א‫' | ||
| + | |- | ||
| + | | | ||
| + | ::We find that if we would have asked for the height of a wall whose base is buried one cubit in the ground and its half, its third and its seventh are above the ground, the height of the whole wall would have been 42 cubits. | ||
| + | |style="text-align:right;"|נמצא אם היינו שואלי' גובה החמה שיסודה שקוע אמה אחת וחצי ושלישי' ושביעי' למעלה למעלה מן הקרקע אז יהיה <s>ש</s> גובהה של כל החומה מ"ב אמות | ||
| + | |- | ||
| + | | | ||
| + | ::Now, we need to learn and evaluate how much it is for 5 cubits, meaning when it is buried 5 cubits in the ground. | ||
| + | |style="text-align:right;"|אבל עכשיו צריכין אנו ללמוד ולהעריך כמה מגיע לה' אמו' פי' שיהיה שקוע ה' אמות בקרקע | ||
| + | |- | ||
| + | | | ||
| + | ::Do the ratio as follows: | ||
| + | |style="text-align:right;"|וכן נעשה הערך | ||
| + | |- | ||
| + | | | ||
| + | ::First, write one cubit, then, slightly far from it, we write 42 cubits, and beneath the one cubit we write the 5 cubits we have asked for, like this: | ||
| + | |style="text-align:right;"|נכתוב תחיל' האמה האחת ואח"כ רחוק ממנו מעט נכתוב מ"ב אמות ותחת האמה האח' נכתוב הה' אמות ששאלנו ויהיה כך | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color: blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |42||1 | ||
| + | |- | ||
| + | | ||5 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |בד||א | ||
| + | |- | ||
| + | | ||ה | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::<span style=color:Green>'''Rule of Three:'''</span> We multiply 5 by 42, meaning: we count how much is 5 times 42. The result is 210. | ||
| + | |style="text-align:right;"|נכפול ה' על מ"ב פי' נמנה כמה עולה ה' פעמי' מ"ב והעולה הוא ר"י | ||
| + | |- | ||
| + | | | ||
| + | ::We divide 210 by 1, meaning: count how many 1 there are in 210. We find that there are 210 times 1 in it and this is the height we asked for. Like this: | ||
| + | ::<math>\scriptstyle{\color{blue}{X=\frac{5\sdot42}{1}=\frac{210}{1}=210}}</math> | ||
| + | |style="text-align:right;"|נחלק ר"י לא'א' פי' נמנה כמה פעמי' א' יש בר"י ונמצא בו ר"י פעמים א' והנה גובה החומה ששאלנו והדמיון כך | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color: blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |2||1||0 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ב||א||‫0 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Here you have another short way how you can know the height of the whole wall, when it is buried 5 cubits in the ground, and its half, its third, and its seventh are up above the ground: | ||
| + | |style="text-align:right;"|וזה לך דרך אחר בקוצר היאך תוכל לידע כמה גובהה של כל החומה כשהיא משוקעת בארץ ה' אמות ולמעלה מן הקרקע היא גבוה החציה ושליש ושביעי‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Take 2 times 5, it is 10; 10 times 3, it is 30; 30 times 7, it is 210. | ||
| + | |style="text-align:right;"|תקח ב' פעמי' ה' הרי י' י' פעמי' ג' הרי ל' פעמי' ז' הרי ר"י | ||
| + | |- | ||
| + | | | ||
| + | ::Or, 2 times 5, it is 10; 10 times 3, it is 30; 30 times 7, it is 210. | ||
| + | ::<math>\scriptstyle{\color{blue}{X=2\sdot5\sdot3\sdot7=10\sdot3\sdot7=30\sdot7=210}}</math> | ||
| + | |style="text-align:right;"|או נלך לדרך זו ב' פעמי' ה' הרי י' יפ"ג ג' הרי ל' לפ"ז הרי ר"י | ||
| + | |- | ||
| + | ! | ||
| + | |||
| + | ==== <span style=color:Green>Joint Purchase Problems - If You Give Me</span> ==== | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:fish|664|mxNb}}3) Another question: there is a fish to sell, and 3 people want to buy it. | ||
| + | :One of them said to his friends: I will give all that I have and each of you will give a half of his and the fish is paid. | ||
| + | :The second said: I will give all that I have and each of you will give one third. | ||
| + | :The third said: I will give all that I have and each of you [will give] a quarter. | ||
| + | :Now it is asked: for how much is the fish being sold and how much does each of them have? | ||
| + | |style="text-align:right;"|<big>ג הא</big> לך שאלה אם אדם אחד אומר לך הנה יש כאן דג אחד למכור והנה ג' <s>פעמי'</s> בני אדם רוצים לקנותו<br> | ||
| + | ואחד מהן אמר לחבירו אתן כל אשר לי וכל אחד מכם יתן החציה ממה שלו ואז יהיה הדג פרוע<br> | ||
| + | והשני אמר אתן כל אשר לי וכל אחד מכם יתן השליש<br> | ||
| + | והשלישי אמר אתן כל אשר לי וכל אחד מכם הרביעי‫'<br> | ||
| + | ועתה שואלי' בכמה רוצי' ליתן הדג או כמה יש לכל אחד ואחד{{#annotend:mxNb}} | ||
| + | |- | ||
| + | | | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle a+\frac{1}{2}\sdot\left(b+c\right)=17\\\scriptstyle b+\frac{1}{3}\sdot\left(a+c\right)=17\\\scriptstyle c+\frac{1}{4}\sdot\left(a+b\right)=17\\\end{cases}</math> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | ::Answer: they want to pay 17 pešuṭim for the fish. | ||
| + | |style="text-align:right;"|<big>תשובה</big> הדג רוצי' ליתן עבור י"ז פשי‫' | ||
| + | |- | ||
| + | | | ||
| + | ::The first has 5 pešuṭim [<math>\scriptstyle{\color{blue}{a=5}}</math>]. | ||
| + | |style="text-align:right;"|והראשון יש לו ה' פשי‫' | ||
| + | |- | ||
| + | | | ||
| + | ::The second has 11 pešuṭim [<math>\scriptstyle{\color{blue}{b=11}}</math>]. | ||
| + | |style="text-align:right;"|והשני י"א פשי‫' | ||
| + | |- | ||
| + | | | ||
| + | ::The third has 13 pešuṭim [<math>\scriptstyle{\color{blue}{c=13}}</math>] | ||
| + | |style="text-align:right;"|והשלישי יש לו י"ג פשי‫' | ||
| + | |- | ||
| + | | | ||
| + | :This is for integers. | ||
| + | |style="text-align:right;"|זהו בשלימי‫' | ||
| + | |- | ||
| + | | | ||
| + | ::But, for fractions: such as if one asks when the fish is bought for 8 pešuṭim, or for 9 pešuṭim , or for 30 pešuṭim , or for 1 pašuṭ, or for whichever amount of money he wish to ask, and for that amount asked: | ||
| + | ::One said: I will give all that I have and you will give a half of yours. | ||
| + | ::The second said: I will give all that I have and you will give one third of yours. | ||
| + | ::The third said: I will give all that I have and you will give a quarter of yours. | ||
| + | ::How much does each of them have, given that this is the amount? | ||
| + | |style="text-align:right;"|אבל השברי' כגון אם ישאל השואל כשהדג נקנה עבור ח' פשי' או ט' פשי' או י"ח פשי' או ל' פשי' או מ' פשי' או איזה סכום שירצה לשאול<br> | ||
| + | ‫<ref>118r</ref>ולאותו סכום ששואל אמר האחד אתן כא"ל ואתם תנו שליש שלכם<br> | ||
| + | והשלישי אמר אתן כא"ל ואתם תנו הד' שלכם<br> | ||
| + | כמה יהיה לכל אחד ואחד ויהיה הסכום שוה | ||
| + | |||
| + | |- | ||
| + | | | ||
| + | :Here I will write you two or three examples, so you can understand everything such as that: | ||
| + | |style="text-align:right;"|והנה אכתוב לך ב' או ג' דמיונות כדי שתוכל להבין כל כיוצא בזה | ||
| + | |- | ||
| + | | | ||
| + | *If the fish is bought for 8 pešuṭim and the first says as above and so does the second and the third | ||
| + | |style="text-align:right;"|אם הדג נקנה עבור ח"פ ואמר הראשון אתן כא"ל ואתם תנו החציה שלכם והשני אמר תנו הג' שלכם והשלישי אמר תנו הרביעי | ||
| + | |- | ||
| + | | | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\begin{cases}\scriptstyle A+\frac{1}{2}\sdot\left(B+C\right)=8\\\scriptstyle B+\frac{1}{3}\sdot\left(A+C\right)=8\\\scriptstyle C+\frac{1}{4}\sdot\left(A+B\right)=8\\\end{cases}}}</math> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | ::The first should have 2 pešuṭim and six parts, of which 17 are a whole unit. | ||
| + | ::<math>\scriptstyle{\color{blue}{A=2+\frac{6}{17}}}</math> | ||
| + | |style="text-align:right;"|צריך שיהא לראשון ב"פ וששה חלקי' שי"ז מהן עושי' חלק שלם | ||
| + | |- | ||
| + | | | ||
| + | ::The second should have 5 pešuṭim and 3 parts, of which 17 are a whole unit. | ||
| + | ::<math>\scriptstyle{\color{blue}{B=5+\frac{3}{17}}}</math> | ||
| + | |style="text-align:right;"|ולשני ה"פ וג' חלקי' שי"ז מהן עושי' חלק שלם | ||
| + | |- | ||
| + | | | ||
| + | ::The third should have 6 pešuṭim and 2 parts, of which 17 are a whole unit. | ||
| + | ::<math>\scriptstyle{\color{blue}{C=6+\frac{2}{17}}}</math> | ||
| + | |style="text-align:right;"|‫[ולשלישי ו' פשיטי' וב' חלקים שי"ז עושין חלק שלם‫] | ||
| + | |- | ||
| + | | | ||
| + | :Now, I will show you the way, by which you will see that it is so: | ||
| + | |style="text-align:right;"|ועתה אראה לך הדרך שתמצא שכן הוא | ||
| + | |- | ||
| + | | | ||
| + | ::<span style=color:Green>'''Rule of Three:'''</span> if it were with integers, the first would have had 5 pešuṭim, as I explained above. Now that it is with fractions and the fish is bought for 8 pešuṭim: | ||
| + | ::<math>\scriptstyle{\color{blue}{5:17=8:A}}</math> | ||
| + | |style="text-align:right;"|הנה אם היה בשלימי' אז היה לראשון ה"פ כמו שפירשתי לעיל<br> | ||
| + | ועתה שהוא בשברי' ועתה כשהדג נקנה בח' פשי‫' | ||
| + | |- | ||
| + | | | ||
| + | ::You should multiply, according to the chapter of multiplication, 8 times 5; it is 40. | ||
| + | |style="text-align:right;"|אתה צריך להטותו בשער הכפל חפ"ה זהו מ‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Then, you should divide it, according to the chapter of division: how many times 17 there are in 40? There are 2 times and six remains, which is less than 17 that is a whole unit. | ||
| + | |style="text-align:right;"|ואח"כ צריך אתה להטותו בשער החילוק ולחלק כמה פעמי' י"ז יש במ' והנה יש ב"פ וששה עודפי' שלא הגיע <s>לששה</s> לי"ז שהן חלק שלם | ||
| + | |- | ||
| + | | | ||
| + | ::We find that the first has 2 pešuṭim and six parts, of which 17 are a whole unit. | ||
| + | ::<math>\scriptstyle{\color{blue}{A=\frac{8\sdot5}{17}=\frac{40}{17}=2+\frac{6}{17}}}</math> | ||
| + | |style="text-align:right;"|נמצא שיש לראשון ב"פ וששה חלקי' שי"ז מהן חלק שלם | ||
| + | |- | ||
| + | | | ||
| + | :Now, we will explain how much does the second have: | ||
| + | |style="text-align:right;"|ועתה נפרש כמה שיש לשיני | ||
| + | |- | ||
| + | | | ||
| + | ::<span style=color:Green>'''Rule of Three:'''</span> if it were with [integers], he would have had 11 pešuṭim. Now that it is with fractions, say as you have said for the first: | ||
| + | |style="text-align:right;"|אם היה בשליש' כמה היו לו י"א<br> | ||
| + | עתה שהוא בשברי' אז תאמר לו כמו שאמרת לראשון | ||
| + | |- | ||
| + | | | ||
| + | ::For the first you have said: "8 times 5". So, for the second say: 8 times 11; the result is 88. | ||
| + | |style="text-align:right;"|פי' לראשון אמרת ח' פ"ה כן תאמר לשני ח"פ י"א והעולה פ"ח | ||
| + | |- | ||
| + | | | ||
| + | ::Then, divide 88 by 17, meaning: how many times 17 there are in 88? There are 5 times 17 in it and 3 remains. | ||
| + | |style="text-align:right;"|ואח"כ תחלוק פ"ח לי"ז י"ז פי' כמה פעמי' י"ז יש בפ"ח ו והנה יש בו ה' פעמי' י"ז וג' עודפי‫' | ||
| + | |- | ||
| + | | | ||
| + | ::We find that the second has 2 pešuṭim and 3 parts, of which 17 are a whole unit. | ||
| + | ::<math>\scriptstyle{\color{blue}{B=\frac{8\sdot11}{17}=\frac{88}{17}=5+\frac{3}{17}}}</math> | ||
| + | |style="text-align:right;"|<s>ג'</s> <sup>נמצא שיש לשני ה"פ וג' חלקי' עודפי'</sup> שי"ז מהן עולין חלק שלם | ||
| + | |- | ||
| + | | | ||
| + | :Do the same for the third: | ||
| + | |style="text-align:right;"|וכן תעשה לשלישי | ||
| + | |- | ||
| + | | | ||
| + | ::<span style=color:Green>'''Rule of Three:'''</span> say: if it were with integers, he would have had 13 pešuṭim. Now that it is in fractions, say: 8 times 13 is 104. | ||
| + | |style="text-align:right;"|ותאמר אם היה בשלימי' היו לו י"ג פשי‫'<br> | ||
| + | ועכשיו שהוא בשברי' אז תאמר לו ח' פעמי' י"ג הרי ק"ד | ||
| + | |- | ||
| + | | | ||
| + | ::Then, [divide] 104 by 17, [meaning]: how many times 17 there are in 104? You find that there are 6 times 17 in it and 2 remains. | ||
| + | |style="text-align:right;"|ואח"כ [תחלק] ק"ד לי"ז י"ז [פי'] כמה פעמי' י"ז יש בק"ד ותמצא בו ו' פעמי' שי"ז [ב'] מהן עודפי‫' | ||
| + | |- | ||
| + | | | ||
| + | ::We find that the third has 6 pešuṭim and 2 parts, of which 17 are a whole unit. | ||
| + | ::<math>\scriptstyle{\color{blue}{C=\frac{8\sdot13}{17}=\frac{104}{17}=6+\frac{2}{17}}}</math> | ||
| + | |style="text-align:right;"|נמצא שיש לשלישי ו"פ וב' חלקי' שי"ז מהן חלק שלם | ||
| + | |- | ||
| + | | | ||
| + | *If the question concerns a fish that is bought for 30 pešuṭim. | ||
| + | |style="text-align:right;"|ואם השאלה מדג הנקנה בל' פשי‫' | ||
| + | |- | ||
| + | | | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\begin{cases}\scriptstyle A+\frac{1}{2}\sdot\left(B+C\right)=30\\\scriptstyle B+\frac{1}{3}\sdot\left(A+C\right)=30\\\scriptstyle C+\frac{1}{4}\sdot\left(A+B\right)=30\\\end{cases}}}</math> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | ::Say: 30 times [5] for the first, and write the result. Then divide it by 17, as I instructed you. | ||
| + | ::<math>\scriptstyle{\color{OliveGreen}{A=\frac{30\sdot5}{17}}}</math> | ||
| + | |style="text-align:right;"|אז תאמר לראשון ל' פעמי' והעולה כתוב ואח"כ תחלקיהו כמו שציויתיך | ||
| + | |- | ||
| + | | | ||
| + | ::Likewise for the second and for the third as above. | ||
| + | |style="text-align:right;"|וכן לשני וכן לשלשי כדלעיל | ||
| + | |- | ||
| + | | | ||
| + | :Now, that I wrote above that the first has 5 pešuṭim, the second 11 and the third 13 in integers, I shall show you the way by which you find that the total is indeed so, that all have 29. | ||
| + | |style="text-align:right;"|ועתה כתבת לעיל שיש לראשון ה' ולשני י"א ולשלישי י"ג בשלימי' הנה אראך הדרך שתמצא ‫<ref>118v</ref>שהכלל כך הוא של כולם יש כ"ט | ||
| + | |- | ||
| + | | | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle X+\frac{1}{2}\sdot\left(Y+Z\right)=a\\\scriptstyle Y+\frac{1}{3}\sdot\left(X+Z\right)=a\\\scriptstyle Z+\frac{1}{4}\sdot\left(X+Y\right)=a\\\end{cases}</math> | ||
| + | ::<math>\scriptstyle{\color{blue}{5+11+13=29}}</math> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | ::Take three numbers such that when you subtract from them a half, a third, and a quarter, you are left with 3; they are: 4, 4 and a half, 6. | ||
| + | |style="text-align:right;"|קח ג' חשבונות כשתסיר מהן החציי' והשליש והרשביעי' וישאר בידך ג' ואילו הן ד'ד' וחצי ו‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Subtract the quarter from 4; [3 remains]. | ||
| + | ::<math>\scriptstyle{\color{blue}{4-\left(\frac{1}{4}\sdot4\right)=3}}</math> | ||
| + | |style="text-align:right;"|תסיר הרביע מן הד' [וישאר ג‫'] | ||
| + | |- | ||
| + | | | ||
| + | ::The third from 4 and a half; [3 remains]. | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(4+\frac{1}{2}\right)-\left[\frac{1}{3}\sdot\left(4+\frac{1}{2}\right)\right]=3}}</math> | ||
| + | |style="text-align:right;"|והשליש מן ד' וחצי [וישאר ג‫'] | ||
| + | |- | ||
| + | | | ||
| + | ::The half from 6; 3 remains from each. | ||
| + | ::<math>\scriptstyle{\color{blue}{6-\left(\frac{1}{2}\sdot6\right)=3}}</math> | ||
| + | |style="text-align:right;"|והחצי מן ו' וישאר ג' מכל ג' אחד ואחד | ||
| + | |- | ||
| + | | | ||
| + | ::This is the denominator, ''those of straying spirit shall come to understanding'' [Isaiah 29, 24] | ||
| + | |style="text-align:right;"|וזה המורה ''{{#annot:Isa29-24|494|frjx}}תועי רוח בינה{{#annotend:frjx}}''‫<ref group=note>ישעיה כט, כד</ref> | ||
| + | |- | ||
| + | | | ||
| + | ::Now, since this denominator [contains parts that are not] integers, for 4 and a half is not an integer: | ||
| + | |style="text-align:right;"|ועתה יען כי אין במור' שלימי' כי ד' וחצי אינו שלם | ||
| + | |- | ||
| + | | | ||
| + | ::Double 4; it is 8. | ||
| + | ::<math>\scriptstyle{\color{blue}{2\sdot4=8}}</math> | ||
| + | |style="text-align:right;"|כפול ד' ויהיה ח‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Double 4 and a half; it is 9. | ||
| + | ::<math>\scriptstyle{\color{blue}{2\sdot\left(4+\frac{1}{2}\right)=9}}</math> | ||
| + | |style="text-align:right;"|כפול ד' וחצי ויהיה ט‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Double 6; it is 12. | ||
| + | ::<math>\scriptstyle{\color{blue}{2\sdot6=12}}</math> | ||
| + | |style="text-align:right;"|כפול <s>י"ב</s> ו' ויהיה י"ב | ||
| + | |- | ||
| + | | | ||
| + | ::The total is 29. | ||
| + | ::<math>\scriptstyle{\color{blue}{8+9+12=29}}</math> | ||
| + | |style="text-align:right;"|ס"ה כ"ט | ||
| + | |- | ||
| + | | | ||
| + | :Yet, we do not know how much is the share of each. Now I shall teach you: | ||
| + | |style="text-align:right;"|ועדיין אין אנו יודעין כמה מגיע לכל אחד לחלקו ועתה אשכילך | ||
| + | |- | ||
| + | | | ||
| + | ::Subtract 2 times 12 from 29; 5 remains and it is the share of the first. | ||
| + | ::<math>\scriptstyle{\color{blue}{X=29-\left(2\sdot12\right)=5}}</math> | ||
| + | |style="text-align:right;"|{{#annot:term|181,1252|0AVC}}הסיר{{#annotend:0AVC}} ב' פעמי' י"ב מכ"ט וישאר ה' והוא חלק של ראשון | ||
| + | |- | ||
| + | | | ||
| + | ::Subtract 2 times 9; 11 remains and it is the share of the second. | ||
| + | ::<math>\scriptstyle{\color{blue}{Y=29-\left(2\sdot9\right)=11}}</math> | ||
| + | |style="text-align:right;"|וכן הסר ב' פעמי' ט' מכ"ט וישאר י"א והוא של שני | ||
| + | |- | ||
| + | | | ||
| + | ::Subtract 2 times 8; 13 remains and it is the share of the third. | ||
| + | ::<math>\scriptstyle{\color{blue}{Z=29-\left(2\sdot8\right)=13}}</math> | ||
| + | |style="text-align:right;"|וכן הסר בפ"ח מכ"ט וישאר י"ג והוא חלק של שלישי | ||
| + | |- | ||
| + | | | ||
| + | ::We find that when the first gives all he has and each of [the others] gives a half, the total is 17. | ||
| + | ::<math>\scriptstyle{\color{blue}{a=X+\frac{1}{2}\sdot\left(Y+Z\right)=17}}</math> | ||
| + | |style="text-align:right;"|נמצא כשהראשון אומר אתן כא"ל וכ"א"מ יתן החציה יהיה ס"ה י"ז | ||
| + | |- | ||
| + | | | ||
| + | ::We find that the fish is bought for 17 pešuṭim and so on. Note well. | ||
| + | |style="text-align:right;"|נמצא שהדג נקנה בי"ז פשי' וכן כולם ודוק | ||
| + | |- | ||
| + | | | ||
| + | :Likewise if the buyers are four, or five and so on endlessly, who wish to buy the fish: take the number of them, i.e. if the buyers are three, take a number such that when you subtract a third, a quarter, and a half, 3 remains; if the buyers are four, take a number such that when you subtract a half, a third, a quarter, and a fifth, 4 remains; and so on. | ||
| + | |style="text-align:right;"|וכן אם הקוני' ד' או ה' עד אין סוף הרוצה לקנות דג קח מניין השוה בכולם פי' או קונין ג' אז קח המניין כשתסיר השליש והרביע וחציי' וישאר ג' כמו שפירשנו או אם הקוני' ד' קח המניין כשתסיר החציי' והשליש והרביעי' והחמישי' וישאר ד' וכן ה' קח המניין כשתסיר חצי של רביעי' חמישי' ששית ונשאר ה' וכן כולם | ||
| + | |- | ||
| + | | | ||
| + | :When the number is not an integer, multiply it so it becomes an integer, then as you did with the integer - if the buyers are three, subtract [the multiple] two times; and if they are four, subtract the multiple three times; and so on. | ||
| + | |style="text-align:right;"|וכשאין המניין בשלימים תכפול אות' שיהא החשבון שלם ואח"כ כעשית החשבון שלם אם הקוני' ג' תסיר ב' פעמי' ואם ד' תסיר ג' פעמי' הכפיל וכן לעולם | ||
| + | |- | ||
| + | !<span style=color:Green>MS P1088, margin:</span> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | *If the buyers are four and the fourth says: I will give all that I have and you will give only a fifth. | ||
| + | |style="text-align:right;"|ואם הקוני' ד' והרביעי אומ' אתן כא"ל וכל אחד יתן רק החומש | ||
| + | |- | ||
| + | | | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle X+\frac{1}{2}\sdot\left(Y+U+V\right)=a\\\scriptstyle Y+\frac{1}{3}\sdot\left(X+U+V\right)=a\\\scriptstyle U+\frac{1}{4}\sdot\left(X+Y+V\right)=a\\\scriptstyle V+\frac{1}{5}\sdot\left(X+Y+U\right)=a\\\end{cases}</math> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | ::Take four numbers: | ||
| + | |style="text-align:right;"|אז קח ד' מנינים | ||
| + | |- | ||
| + | | | ||
| + | ::Subtract its half from it and 4 remains; it is 8. | ||
| + | ::<math>\scriptstyle{\color{blue}{8-\left(\frac{1}{2}\sdot8\right)=4}}</math> | ||
| + | |style="text-align:right;"|אחר תסיר ממנו חצי וישאר ד' וזהו ח‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Subtract its third and 4 remains; it is 6. | ||
| + | ::<math>\scriptstyle{\color{blue}{6-\left(\frac{1}{3}\sdot6\right)=4}}</math> | ||
| + | |style="text-align:right;"|ואחר תסיר ממנו שליש וישאר ד' זהו ו‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Subtract its quarter and 4 remains; it is 5 and a third. | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(5+\frac{1}{3}\right)-\left[\frac{1}{4}\sdot\left(5+\frac{1}{3}\right)\right]=4}}</math> | ||
| + | |style="text-align:right;"|ואחר תסיר ממנו רביעי וישאר ד' זהו ה' ושלישי | ||
| + | |- | ||
| + | | | ||
| + | ::Subtract its fifth and 4 remains; it is 5. | ||
| + | ::<math>\scriptstyle{\color{blue}{5-\left(\frac{1}{5}\sdot5\right)=4}}</math> | ||
| + | |style="text-align:right;"|ואחר תסיר ממנו החומש וישאר ד' זהו ה‫' | ||
| + | |- | ||
| + | | | ||
| + | ::One should take these numbers three times and then the third will become an integer: | ||
| + | |style="text-align:right;"|וצריך לקח' זה החשבונות ג' פעמים ואז יהא השליש שלם | ||
| + | |- | ||
| + | | | ||
| + | ::Now, take 3 times 8; it is 24. | ||
| + | ::<math>\scriptstyle{\color{blue}{3\sdot8=24}}</math> | ||
| + | |style="text-align:right;"|ועתה קח ח' ג' פעמי' זהו ד"ב | ||
| + | |- | ||
| + | | | ||
| + | ::Take 3 times 6; it is 18. | ||
| + | ::<math>\scriptstyle{\color{blue}{3\sdot6=18}}</math> | ||
| + | |style="text-align:right;"|קח ו' ג"פ זהו ח"א | ||
| + | |- | ||
| + | | | ||
| + | ::Take 3 times 5 and a third; it is 16. | ||
| + | ::<math>\scriptstyle{\color{blue}{3\sdot\left(5+\frac{1}{3}\right)=16}}</math> | ||
| + | |style="text-align:right;"|קח ה' ושלי' ג"פ זהו ו"א | ||
| + | |- | ||
| + | | | ||
| + | ::Take 3 times 5; it is 15. | ||
| + | ::<math>\scriptstyle{\color{blue}{3\sdot5=15}}</math> | ||
| + | |style="text-align:right;"|קח ה' ג"פ זהו ה"א | ||
| + | |- | ||
| + | | | ||
| + | ::The total sum is 73. | ||
| + | ::<math>\scriptstyle{\color{blue}{24+18+16+15=73}}</math> | ||
| + | |style="text-align:right;"|סך הכל ג"ז | ||
| + | |- | ||
| + | | | ||
| + | ::Now, subtract 3 times 24 from 73; 1 remains. We find that Reuven [has] 1. | ||
| + | ::<math>\scriptstyle{\color{blue}{X=73-\left(3\sdot24\right)=1}}</math> | ||
| + | |style="text-align:right;"|ועתה הסר ג"פ ד"ב מג"ז וישאר א' נמצא ש[......] ראובן א‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Subtract 3 times 18 from 73; 19 remains. It is Shimon's amount. | ||
| + | ::<math>\scriptstyle{\color{blue}{Y=73-\left(3\sdot18\right)=19}}</math> | ||
| + | |style="text-align:right;"|והסר ג"פ ח"א מג"ז וישאר ט"א זה סך שמעון | ||
| + | |- | ||
| + | | | ||
| + | ::Subtract 3 times 16 from 73; 25 remains. It is Levi's amount. | ||
| + | ::<math>\scriptstyle{\color{blue}{U=73-\left(3\sdot16\right)=25}}</math> | ||
| + | |style="text-align:right;"|והסר ו"א ג"פ מג"ז וישאר ה"ב זהו סך לוי | ||
| + | |- | ||
| + | | | ||
| + | ::Subtract 3 times 15 from 73; 28 remains. It is Yehuda's amount. | ||
| + | ::<math>\scriptstyle{\color{blue}{V=73-\left(3\sdot15\right)=28}}</math> | ||
| + | |style="text-align:right;"|והסר ה"א ג"פ מג"ז וישאר ח"ב זהו סך יודא | ||
| + | |- | ||
| + | | | ||
| + | ::The total sum is 73 and the fish was bought for 37. | ||
| + | |style="text-align:right;"|הכל ג"ז והדג נקנה בעד ז"ג | ||
| + | |- | ||
| + | | | ||
| + | :Examine and you will find [that it is true] | ||
| + | |style="text-align:right;"|ודוק ותמצא | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:a thing|664|92Y0}}4) Question: three people - Reuven, Shimon, and Levi – are going to the market to buy something. | ||
| + | :Reuven said: I will give all that I have and each of you will give one third of what is in his pocket. | ||
| + | :Shimon said: I will give all that I have and each of you will give a sixth of what is in his pocket. | ||
| + | :Levi said: I will give all that I have and each of you will give a ninth of what is in his pocket | ||
| + | |style="text-align:right;"|<big>ד הנה</big> ג' אנשים הולכי' על השוק ראובן שמעון ולוי לקנות דבר<br> | ||
| + | והנה ראובן אמר אתן כל אשר לי וכל אחד מכם יתן הג' שבכיסו<br> | ||
| + | ושמעון אמר אתן כא"ל וכא"מ יתן השישי' שבכיסו<br> | ||
| + | ולוי אמר אתן כא"ל וכא"מ יתן התשיעי' שבכיסו{{#annotend:92Y0}} | ||
| + | |- | ||
| + | | | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle X+\frac{1}{3}\sdot\left(Y+Z\right)=a\\\scriptstyle Y+\frac{1}{6}\sdot\left(X+Z\right)=a\\\scriptstyle Z+\frac{1}{9}\sdot\left(X+Y\right)=a\\\end{cases}</math> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | ::Now I shall teach you the way: | ||
| + | |style="text-align:right;"|ועתה אשכילך הדרך | ||
| + | |- | ||
| + | | | ||
| + | ::You have three people: Reuven, Shimon, and Levi. Reuven asks a third, Shimon a sixth, and Levi a ninth. | ||
| + | |style="text-align:right;"|הנה יש לך ג' אנשי' ראובן שמעון ולוי ראובן שואל שליש ושמעון ששית ולוי תשיעי' נגדו | ||
| + | |- | ||
| + | | | ||
| + | ::Write three numerals for their demands, as follows: | ||
| + | |style="text-align:right;"|כתוב ג' אותיו' לפי שאילת' כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color: blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |9||6||3 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ט||ו||ג | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Take the least number, such that when you subtract a third from it, 3 remains for the members of the problem; it is 4 and a half. | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(4+\frac{1}{2}\right)-\left[\frac{1}{3}\sdot\left(4+\frac{1}{2}\right)\right]=3}}</math> | ||
| + | |style="text-align:right;"|וקח חשבון המועט תקח ממנו השלישי' וישאר ג' נגד בני החיד' זהו ד' וחצי | ||
| + | |- | ||
| + | | | ||
| + | ::Write the 4 and a half beneath the 3, like this: | ||
| + | |style="text-align:right;"|וכותבו תחת הג' הד' וחצי כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color: blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |9||6||3 | ||
| + | |- | ||
| + | | || ||4 | ||
| + | |- | ||
| + | | || ||half | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ט||ו||ג | ||
| + | |- | ||
| + | | || ||ד | ||
| + | |- | ||
| + | | || ||וחצי | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Take the least number, such that when you subtract a sixth from it, 3 remains for the members of the problem; it is 3 3-fifths. | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(3+\frac{3}{5}\right)-\left[\frac{1}{6}\sdot\left(3+\frac{3}{5}\right)\right]=3}}</math> | ||
| + | |style="text-align:right;"|וקח חשבון המועט שתקח ממנו הששית ‫<ref>119r</ref>וישאר ג' כנגד בני החידה וזהו ג' וג' חומשיהו | ||
| + | |- | ||
| + | | | ||
| + | ::Write it beneath the 6, like this: | ||
| + | |style="text-align:right;"|ותכתבהו תחת הו' כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color: blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |9||6||3 | ||
| + | |- | ||
| + | | ||3||4 | ||
| + | |- | ||
| + | | ||⅗||half | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ט||ו||ג | ||
| + | |- | ||
| + | | ||ג||ד | ||
| + | |- | ||
| + | | ||ג"ה||וחצי | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Take the least number, such that when you subtract a ninth from it, 3 remains for the members of the problem; it is 3 and 3-eighths. | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(3+\frac{3}{8}\right)-\left[\frac{1}{9}\sdot\left(3+\frac{3}{8}\right)\right]=3}}</math> | ||
| + | |style="text-align:right;"|ותקח חשבון המועט שכשתיקח התשיעי' וישאר ג' כנגד בני החידה זהו ג' וג' שמניות | ||
| + | |- | ||
| + | | | ||
| + | ::Write it also beneath the 9, like this: | ||
| + | |style="text-align:right;"|ותכתבהו ג"כ תחת הט' כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color: blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |9||6||3 | ||
| + | |- | ||
| + | |3||3||4 | ||
| + | |- | ||
| + | |⅜||⅗||half | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ט||ו||ג | ||
| + | |- | ||
| + | |ג||ג||ד | ||
| + | |- | ||
| + | |ג"ח||ג"ה||וחצי | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | :Now, take the least number in which you find an integer for these fractions. | ||
| + | |style="text-align:right;"|ועתה קח חשבון המועט שתמצא בו חשבון שלם נגד אותן חלקי' שברים שיש לך | ||
| + | |- | ||
| + | | | ||
| + | ::Such as a half, a fifth, and an eighth: take a number in which you find a half that is an integer; it is 2. | ||
| + | |style="text-align:right;"|כגון חצי חמישי' שמיני' עתה קח חשבון שתמצא בו חצי שלם זהו ב‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Take the least number in which you find a fifth that is an integer; it is 5. | ||
| + | |style="text-align:right;"|וקח חשבון שתמצא חמישי' שלם זהו ה‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Take the least number in which you find an eighth that is an integer; it is 8. | ||
| + | |style="text-align:right;"|וג"כ קח חשבון המועט שתמצא בו שמיני' שלם זהו ח‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Multiply 2 by 5; it is ten, like this: | ||
| + | ::<math>\scriptstyle{\color{blue}{2\sdot5=10}}</math> | ||
| + | |style="text-align:right;"|תכפול ב' על ה' ויהיה עשרה כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color: blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |1||0 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |א||‫0 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::You find that both are integers in it: a half that is an integer and a fifth that is an integer. | ||
| + | |style="text-align:right;"|בזה תמצא שניהם חצי שלם וחמישי' שלם | ||
| + | |- | ||
| + | | | ||
| + | ::Now, multiply and say: 10 times 4, which is a half of 8; it is 40, like this: | ||
| + | ::<math>\scriptstyle{\color{blue}{10\sdot\left(\frac{1}{2}\sdot8\right)=10\sdot4=40}}</math> | ||
| + | |style="text-align:right;"|עתה תכפול ותאמר יפ"ד דהיינו חצי ח' זהו מ' כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color: blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |4||0 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ד||‫0 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::You find in it a half that is an integer, a fifth that is an integer, and an eighth that is an integer. | ||
| + | |style="text-align:right;"|בזה תמצא חצי שלם חמישי' שלם שמיני' שלם | ||
| + | |- | ||
| + | | | ||
| + | ::Check: to [multiply] 8 by ten, since the fraction is 3-eighths; then the product were like this: | ||
| + | ::<math>\scriptstyle{\color{blue}{10\sdot\sdot8=80}}</math> | ||
| + | |style="text-align:right;"|ובדו' לפכול ה' עם העשר' נגד חלקי שברי' דהן ג' שמי' ואז היה עולה כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color: blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |8||0 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ח||‫0 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::But, you would find more than you need, for in 40 you will find the calculation as in 80. | ||
| + | ::<span style=color:Green>Least common multiple: LCM(2,5,8) = 40</span> | ||
| + | |style="text-align:right;"|אך היית מצוא יותר מצרכך כי כמה תמצא חשבון כמו ב"פ | ||
| + | |- | ||
| + | | | ||
| + | ::Always take the least number, ''If you grasped many, you did not grasp anything; if you grasped few, you grasped something'' [Talmud, Sukkah, 5a]. | ||
| + | |style="text-align:right;"|ולעולם קח חשבון המועט ''תפשת מרובה לא תפשת תספת מועט תפסת''‫<ref group=note>בבלי, מועד, סוכה, דף ה, א גמרא</ref> | ||
| + | |- | ||
| + | | | ||
| + | ::Now multiply what is written beneath each by 40: | ||
| + | |style="text-align:right;"|ועתה כפול המ' על שכתוב תחת כל אחד | ||
| + | |- | ||
| + | | | ||
| + | ::You find 4 and a half beneath Reuven. Multiply 40 by 4 and a half; the result is like this: | ||
| + | ::<math>\scriptstyle{\color{blue}{40\sdot\left(4+\frac{1}{2}\right)=180}}</math> | ||
| + | |style="text-align:right;"|הנה מצאת תחת ראובן ד' וחצי כפול 0'ד' על ד' וחצי ויעלה כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color: blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |1||8||0 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |א||ח||‫0 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Multiply what is written beneath Levi, i.e. 3 and 3-fifths, by 40; the result is like this: | ||
| + | ::<math>\scriptstyle{\color{blue}{40\sdot\left(3+\frac{3}{5}\right)=144}}</math> | ||
| + | |style="text-align:right;"|וכפול 0"ד על שכתוב תחת שמעון דהיינו ג' וג' חומשי' ויעלה כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color: blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |1||4||4 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |א||ד||ד | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Multiply what is written beneath Shimon, i.e. 3 and 3-eighths, by 40; it is like this: | ||
| + | ::<math>\scriptstyle{\color{blue}{40\sdot\left(3+\frac{3}{8}\right)=135}}</math> | ||
| + | |style="text-align:right;"|וכפול 0'ד' על שכתוב תחת לוי דהיינו ג' וג' שמיניו' ויעלה כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color: blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |1||3||5 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |א||ג||ה | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Sum up these three numbers; the sum is as follows: | ||
| + | ::<math>\scriptstyle{\color{blue}{180+144+135=459}}</math> | ||
| + | |style="text-align:right;"|עת' חבר אילו ג' חשבונות יחד ויעלה כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color: blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |4||5||9 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ד||ה||ט | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::This is the denominator of the problem. | ||
| + | |style="text-align:right;"|וזהו המורה את החידה | ||
| + | |- | ||
| + | | | ||
| + | ::Double 180; the result is 360. Subtract it from the denominator of the problem, which is 459; you are left with 99, and so is Reuven's money. | ||
| + | ::<math>\scriptstyle{\color{blue}{X=459-\left(2\sdot180\right)=459-360=99}}</math> | ||
| + | |style="text-align:right;"|כפול 0ח"א ב' פעמי' ויעלה 0ו"ג ו{{#annot:term|181,2201|zXtY}}נכיהו מן{{#annotend:zXtY}} המורה את החידה שהוא טה"ד ויוותר לך ט"ט כך מעות יש לראובן | ||
| + | |- | ||
| + | | | ||
| + | ::Then, double 144, which is the double of Shimon; the result is 288. Subtract it from the denominator; you are left with 171, and so is Shimon's money. | ||
| + | ::<math>\scriptstyle{\color{blue}{Y=459-\left(2\sdot144\right)=459-288=171}}</math> | ||
| + | |style="text-align:right;"|ואח"כ כפול ב"פ דד"א והוא הייה כפול לשמעון ויעלה חח"ב נכיהו מן המורה ויוותר לך אז"א כך מעות יש לשמעון | ||
| + | |- | ||
| + | | | ||
| + | ::Then, double 135, which is the double of Levi; the result is 270. Subtract it from the denominator; you are left with 189, and so is Levi's money. | ||
| + | ::<math>\scriptstyle{\color{blue}{Z=459-\left(2\sdot135\right)=459-270=189}}</math> | ||
| + | |style="text-align:right;"|ואח"כ כפול ב"פ הג"א שהוא כפול לוי ויעלה 0ז"ב נכיהו מן המורה ויוותר לך טח"א כך מעות יש ללוי | ||
| + | |- | ||
| + | | | ||
| + | ::Now you have found it all: Reuven has 99, Shimon has 171, Levi has 189. | ||
| + | |style="text-align:right;"|עתה מצאת הכל לראובן ט'ט' ולשמעון אז"א וללוי טח"א | ||
| + | |- | ||
| + | | | ||
| + | :The one who understands will understand. | ||
| + | |style="text-align:right;"|והמבין יבין | ||
| + | |- | ||
| + | ! | ||
| + | |||
| + | ==== <span style=color:Green>Find a Quantity Problems - Whole from Parts</span> ==== | ||
| + | | | ||
| + | |- | ||
| + | !<span style=color:Green>Tree</span> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:tree|650|tHGB}}5) Question: a tree - its third, its fifth, and its ninth are planted in the soil and 10 cubits remain up above the ground, how long is it? | ||
| + | :<math>\scriptstyle\frac{1}{3}X+\frac{1}{5}X+\frac{1}{9}X+10=X</math> | ||
| + | |style="text-align:right;"|<big>ה אילן</big> התקוע בארץ שלישיתו חמישיתו ותשיעיתו ונותר למעלה מן הארץ י' אמות כמה הית<s>ת</s> תחילתו{{#annotend:tHGB}} | ||
| + | |- | ||
| + | | | ||
| + | ::Here is the diagram that you arrange. Write like that according to the fractions you asked for: | ||
| + | |style="text-align:right;"|והא לך דמות אשר תעריך כתוב כזה דהיינו נגד החלקי' ששאלת | ||
| + | |- | ||
| + | | | ||
| + | ::Now, put your mind to find the least number, in which the fractions you asked for are found: a third that is an integer, a fifth that is an integer, and a ninth that is an integer. | ||
| + | |style="text-align:right;"|עתה שים דעתך ‫<ref>119v</ref>למצא חשבון המועט שנמצא בו החלקי' ששאלת שליש שלם חמישי' שלם תשיעי' שלם | ||
| + | |- | ||
| + | | | ||
| + | ::Say as follows: 3 times 5 is 15; 9 times 15 is 135. | ||
| + | ::<math>\scriptstyle{\color{blue}{3\sdot5\sdot9=15\sdot9=135}}</math> | ||
| + | |style="text-align:right;"|וכה תאמר ג"פ ה' היינו ה"א ט' פעמי' ה"א יעלה הג"א | ||
| + | |- | ||
| + | | | ||
| + | ::But, you do not need that much, as you find them in 45, which is a third of 135. | ||
| + | ::<span style=color:Green>Least common multiple: LCM(3,5,9) = 45</span> | ||
| + | |style="text-align:right;"|ואינך צריך כ"כ כי תמצאנה בה"ד שהו שליש מן הג"א | ||
| + | |- | ||
| + | | | ||
| + | ::Take the least and not the greater. | ||
| + | |style="text-align:right;"|קח המועט ולא המרובה | ||
| + | |- | ||
| + | | | ||
| + | ::Subtract all the fractions you asked for, i.e. a third, a fifth, and a ninth, from 45. | ||
| + | |style="text-align:right;"|תחסור מן ה"ד כל החלקי' ששאלת דהיינו שלישי' חמישית תשיעי‫' | ||
| + | |- | ||
| + | | | ||
| + | ::A third of 45 is 15; a fifth is 9; a ninth is 5; subtract all of them from the denominator, which is 45; 16 remains. | ||
| + | |style="text-align:right;"|שליש ה"ד ה"א חמישי' ט' תשיעי' ה' תסרם מן המורה שהיה ה"ד ונותר ו"א | ||
| + | |- | ||
| + | |colspan="2"| | ||
| + | ::<math>\scriptstyle{\color{blue}{45-\left(\frac{1}{3}\sdot45\right)-\left(\frac{1}{5}\sdot45\right)-\left(\frac{1}{9}\sdot45\right)=45-15-9-5=16}}</math> | ||
| + | |- | ||
| + | | | ||
| + | ::<span style=color:Green>'''Rule of Three:'''</span> Now, multiply the cubits that remain above the ground, which are 10, by the denominator that is 45; the result is 450. | ||
| + | |style="text-align:right;"|עתה כפול האמות הנותרי' למעלה מן הארץ והם 0"א על המורה שהיה ה"ד ויעלה 0ה"ד | ||
| + | |- | ||
| + | | | ||
| + | ::Divide it by the remaining 16, according to the chapter of division, meaning how many times 16 there are in 450; the result is 28 and 2 parts of 16 of the whole. | ||
| + | ::<math>\scriptstyle{\color{blue}{X=\frac{10\sdot45}{16}=\frac{450}{16}=28+\frac{2}{16}}}</math> | ||
| + | |style="text-align:right;"|עתה [חלוק ממנו]‫<ref>M.: החלק</ref> ו"א הנותרי' [בשער החילוק פי' כמה פעמים יש ו"א ב0'ה'ד'] ויעלה החילוק ח"ב וב' חלקי' שו"א מהן עושי' חלק שלם | ||
| + | |- | ||
| + | | | ||
| + | :So, the whole tree is 28 cubits and 2 parts of 16 of the whole. | ||
| + | |style="text-align:right;"|וכן כל האילן ח"ב אמו' וב' חלקי' שו"א מהן עושין חלק שלם | ||
| + | |- | ||
| + | !<span style=color:Green>Lance</span> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:lance|650|NtSR}}6) Question: a lance - one sticks a half of the whole lance in the ground, then another comes and raises a third of the whole lance, another one comes and sticks a quarter of the whole lance, and one more comes and raises a sixth of the whole lance. After lowering the high and raising the low, 11 cubits remain above the ground. How many cubits are the length of the whole lance? | ||
| + | :<math>\scriptstyle\frac{1}{2}-\frac{1}{3}X+\frac{1}{4}X-\frac{1}{6}X+11=X</math> | ||
| + | |style="text-align:right;"|<big>ו' הנה</big> רומח לפניך ובא אחד ותוקעו בארץ חציו של כל הרומח ובא אחד והגביה שלישיתו של כל הרומח ובא אחד ותוקעו רביעיתו של כל הרומח ובא אחד והגביה שישיתו של כל הרומח ולאחר מן הארץ הגבהה וזה הגביה הנמוך נותר למעלה מן הארץ א'א' אמות כמה אמות ארכו של כל הרומח{{#annotend:NtSR}} | ||
| + | |- | ||
| + | | | ||
| + | ::Here is the way: | ||
| + | |style="text-align:right;"|וזה לך הדרך | ||
| + | |- | ||
| + | | | ||
| + | ::Write for the parts that you asked - 2 for the half, 3 for the third, 4 for the quarter, and 6 for the 6. | ||
| + | |style="text-align:right;"|כתוב נגד החלקי' ששאלת נגד החצי ב' נגד השליש ג' נגד רביעי' ד' נגד הששית ו‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Write the remaining 11 cubits above, like this: | ||
| + | |style="text-align:right;"|וכתוב עליו א'א' אמות הנותרי' כזה | ||
| + | |- | ||
| + | | | ||
| + | ::Take the least number in which you find them, i.e. 12 and this is the denominator. | ||
| + | |style="text-align:right;"|וקח החשבון המועט שתמצא בו אילו שלם דהיינו ב"א וזה המורה | ||
| + | |- | ||
| + | | | ||
| + | ::Subtract a half from 12; 6 remains. | ||
| + | |style="text-align:right;"|הסיר מב"א החציה וישאר ו‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Add one-third of 12 to 6, i.e. 4; it is 10. | ||
| + | |style="text-align:right;"|הוסיף השליש מב"א על הו' [דהיינו ד'] ויהיה 0"א | ||
| + | |- | ||
| + | | | ||
| + | ::Subtract one-quarter of 12, i.e. 3, take it from 10; 7 remains. | ||
| + | |style="text-align:right;"|הסיר הרביע מב"א [דהיינו ג'] מ0"א וישאר ז‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Add one-sixth of 12, i.e. 2, to 7; it is 9. | ||
| + | |style="text-align:right;"|הוסיף הששית [של]‫<ref>M:עולה</ref> ב"א [דהיינו ב'] על ז' ויהיה ט‫' | ||
| + | |- | ||
| + | |colspan="2"| | ||
| + | ::<math>\scriptstyle{\color{blue}{12-\left(\frac{1}{2}\sdot12\right)+\left(\frac{1}{3}\sdot12\right)-\left(\frac{1}{4}\sdot12\right)+\left(\frac{1}{6}\sdot12\right)=6+4-3+2=10-3+2=7+2=9}}</math> | ||
| + | |- | ||
| + | | | ||
| + | ::Write the 9 on another side, ''And your eyes shall see your Teacher'' [Isaiah 20, 30]. | ||
| + | |style="text-align:right;"|אות' [ט'] כתוב בצד אחר ''{{#annot:Isa30-20|494|Kj7t}}ויהיו עיניך רואות את פני מוריך{{#annotend:Kj7t}}''‫<ref group=note>ישעיהו ל, כ</ref> שהוא ב"א | ||
| + | |- | ||
| + | | | ||
| + | ::<span style=color:Green>'''Rule of Three:'''</span> Multiply it by the 11 that remain above the ground; the result is this: | ||
| + | |style="text-align:right;"|וכפול עליו הא"א הנותרי' למעלה מן הארץ ויעלה כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color: blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |1||3||2 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |style="text-align:right;"|א||ג||ב | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Divide it according to the chapter of division by what remains from the denominator after you have subtracted and added all the parts, which is 9. How many times 9 there are in 135? 14 times and 6 are left that do not reach 9. | ||
| + | |style="text-align:right;"|תחלוק ממנו [בשער החילוק] מה שנותר לך מן המורה שלאחר שחסרת והוסף כל החלקי' זהו ט' כמה פעמים ט' יש בבג"א זהו ד"א פעמי' ונותרי' ו' שאינ' מגיעי' לכלל ט‫' | ||
| + | |- | ||
| + | | | ||
| + | ::So, the length of the lance is 14 cubits and 6 parts of 9 of a whole cubit. | ||
| + | ::<math>\scriptstyle{\color{blue}{X=\frac{11\sdot12}{9}=\frac{132}{9}=14+\frac{6}{9}}}</math> | ||
| + | |style="text-align:right;"|וכן אורך הרומח ד"א אמו' ו' חלקי' שט' מהן עולין חלק שלם דהיינו אמה | ||
| + | |- | ||
| + | |This is the rule - when you say: add to it one-third, or one-fifth, or one-quarter, or as much as you want and it is so and so, how much was it originally? | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{X+\frac{1}{a}X=b}}</math> | ||
| + | |style="text-align:right;"|זה הכלל כשתאמר שים שליש עליו או חומש או רביע או כל מה שתרצה ויהיה כך וכך כמה היה בתחילה | ||
| + | |- | ||
| + | |I will show you the way to proceed: | ||
| + | |style="text-align:right;"|אראה לך הדרך אשר תלך בה | ||
| + | |- | ||
| + | |Always subtract from the specified number a fraction [whose denominator is smaller by one than] what you intended to add to the unknown number. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{X=b-\left(\frac{1}{a+1}\sdot b\right)}}</math> | ||
| + | |style="text-align:right;"|‫<ref>120r</ref>לעולם הסר מן החשבון המפורשי' חלק אחד יותר מחשבון הנעלם שדעתך לצרף עליו | ||
| + | |- | ||
| + | | | ||
| + | :*For instance, if you say: add to it one-third and it becomes 5, how much was the specific number originally? | ||
| + | ::<math>\scriptstyle{\color{blue}{X+\frac{1}{3}X=5}}</math> | ||
| + | |style="text-align:right;"|כגון אם תאמר עשה שליש עליו ויהיה ה' זהו המפורש כמה היה בתחילה | ||
| + | |- | ||
| + | | | ||
| + | ::Subtract a quarter from the specified 5, [whose denominator is smaller by one than] what you intended to add to it, since you intended to add a third and this is a quarter. Subtract a quarter from 5; the remainder is the required. | ||
| + | ::<math>\scriptstyle{\color{blue}{X=5-\left(\frac{1}{3+1}\sdot5\right)=5-\left(\frac{1}{4}\sdot5\right)}}</math> | ||
| + | |style="text-align:right;"|אז הסר מן <s>החשבון</s> הה' המפורש רביעי' זהו חלק אחד יותר ממה שבדעתך לצרף עליו כי היה בדעת' לצרף שליש וזהו רבי' תסי' מן [ה'] הרבי' אז הנשא' הוא המבוקש | ||
| + | |- | ||
| + | | | ||
| + | ::When you add to it a third the result is 5. | ||
| + | |style="text-align:right;"|וכשתעשה עליו שליש יהיה ה‫' | ||
| + | |- | ||
| + | | | ||
| + | :*Or, if you say: add to it one-seventh and it becomes 3, [how much was] the specific number [originally]? | ||
| + | ::<math>\scriptstyle{\color{blue}{X+\frac{1}{7}X=3}}</math> | ||
| + | |style="text-align:right;"|או אם תאמר עשה שביעי' עליו ויהיה ג' זהו ג' המפורש | ||
| + | |- | ||
| + | | | ||
| + | ::Subtract an eighth from the specified 3; what remains is the original number. | ||
| + | ::<math>\scriptstyle{\color{blue}{X=3-\left(\frac{1}{7+1}\sdot3\right)=3-\left(\frac{1}{8}\sdot3\right)}}</math> | ||
| + | |style="text-align:right;"|אז הסר מג' המפור' שמי' ומה שנשאר הוא הסכום מה שהיה בתחילה | ||
| + | |- | ||
| + | | | ||
| + | ::When you want to know if you have calculated correctly: add the seventh to the remainder and it will be 3. | ||
| + | |style="text-align:right;"|ואת"ל אם אמת חשבת צרוף שביעי' על הנשאר ויהיה ג‫' | ||
| + | |- | ||
| + | |And so on do as I showed you. | ||
| + | |style="text-align:right;"|וכן לעולם כשאר <s>אב</s> הראיתיך | ||
| + | |- | ||
| + | |But, if you wish to say: subtract one-third and so and so remains, or subtract as much as you want and so and so remains, how much was it originally? | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{X-\frac{1}{a}X=b}}</math> | ||
| + | |style="text-align:right;"|אבל אם ת"ל כשתקח שליש ממנו וישאר כך וכך או תיקח ממנו כל מה שתרצה וישאר כך וכך כמה שהיה בתחילה | ||
| + | |- | ||
| + | |Divide the remaining number by a [denominator] smaller by one than that of the fraction you subtract, then add the fraction to the remainder, and you will find how much it was originally. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{X=b+\left(\frac{1}{a-1}\sdot b\right)}}</math> | ||
| + | |style="text-align:right;"|אז תחלק <s>חלק</s> <sup>חשבון</sup> הנשאר לחלק אחת פחות ממה שחסרת ממנו וחלק אחד מן החלקי' צרוף עם זה הנשאר ותמצא כמה שהיה בתחילה | ||
| + | |- | ||
| + | ! | ||
| + | |||
| + | ==== <span style=color:Green>Shared Work Problems - Filling/Draining a Vessel</span> ==== | ||
| + | |||
| + | | | ||
| + | |- | ||
| + | !<span style=color:Green>Well</span> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:well|625|gjwa}}7) Question: Here is a well whose water is flowing into it through 3 pipes and draining out of it through 2 pipes. | ||
| + | :Through one of the 3 pipes, from which the water flows into it, the well is filled in a sixth of a day, if the water does not drain out from it. | ||
| + | :Through the second pipe, from which [the water] flows into it, the well is filled in a fifth of a day, if the water does not drain out from it. | ||
| + | :Through the third pipe, from which [the water] flows into it, the well is filled in a quarter of a day. | ||
| + | :The two pipes that are draining the well: through one of them, the well is drained in a half of a day, if the water does not flow into it; through the second pipe, the well is drained in a third of a day. | ||
| + | :When the filling pipes are filling the well with water and the draining pipes are draining it all at once, how long will it take the well to be filled? | ||
| + | :<math>\scriptstyle6X+5X+4X-2X-3X=1</math> | ||
| + | |style="text-align:right;"|<big>ז' שאלה</big> הנה לך בור שמימיו נכנסי' בו בג' קני' ויוצאי' ממנו דרך ב' קני‫'<br> | ||
| + | ומהאח' מג' קנים שמימיו נכנסי' בו היה הבור מתמלא מששית היום אם לא היו יוצאין מן הבור מים כלל<br> | ||
| + | ומן הקנה השני שנכנס בו היה הבור מתמלא אם לא יוצאין ממנו בחמישי' היום<br> | ||
| + | ומן הקנה השלישי' שנכנס בו היה הבור מתמלא ממנו ברביעי' היום<br> | ||
| + | וב' קני' המריקי' הבור מן האחד נתרוקן הבור כשאין נכנסי' בו שום מים בחצי היום<br> | ||
| + | ומקנה שני נתרוקן הבור בשליש היום<br> | ||
| + | וכשאילו הקני' מכניסי' מים ממלאי' את הבור יחד וב' קני' המריקי' מריקי' יחד בכמה הבור מתמלא כך כשאילו מריקי' ואילו ממלאין{{#annotend:gjwa}} | ||
| + | |- | ||
| + | | | ||
| + | ::Answer: it is filled in a tenth of a day. | ||
| + | |style="text-align:right;"|תשובה בעשירי' היום מתמלא | ||
| + | |- | ||
| + | | | ||
| + | ::I will teach you the way how: | ||
| + | |style="text-align:right;"|ואשכילך הדרך כיצד | ||
| + | |- | ||
| + | | | ||
| + | ::The first pipe that fills the well in a sixth of a day can fill six wells in one day. | ||
| + | |style="text-align:right;"|קנה האחד שממלא הבור בששי' היום יכול למלא ששה בורות ביו' אחד | ||
| + | |- | ||
| + | | | ||
| + | ::The second [pipe] that fills [the well] in a fifth of a day can fill five wells in one day. | ||
| + | |style="text-align:right;"|והשני שממלא הבור בחמישי' היום יכול למלא חמשה בורות ביום אחד | ||
| + | |- | ||
| + | | | ||
| + | ::The third [pipe] that fills [the well] in a quarter of a day can fill four wells in one day. | ||
| + | |style="text-align:right;"|והשלישי שמתמלא הבור ברביעי' היום יכול למלא ד' בורו' ביום אחד | ||
| + | |- | ||
| + | | | ||
| + | ::Hence, these three pipes would have filled 15 wells in one day, had the two pipes not drained. | ||
| + | ::<math>\scriptstyle{\color{blue}{6+5+4=15}}</math> | ||
| + | |style="text-align:right;"|הרי ט"ו בורות שג' קני' הללו ממלאי' ביום אחד אם ‫<ref>120v</ref>לא היו אלו ב' קני' מריקי‫' | ||
| + | |- | ||
| + | | | ||
| + | ::How long will it take them to drain: | ||
| + | |style="text-align:right;"|ובכמה הן מריקי‫' | ||
| + | |- | ||
| + | | | ||
| + | ::The first [pipe] that drains [the well] in half a day drains two [wells] in one day. | ||
| + | |style="text-align:right;"|האחד המריק' בחצי היום ממריקי' ב' ליום אחד | ||
| + | |- | ||
| + | | | ||
| + | ::The second [pipe] that drains [the well] in a third of a day drains three [wells] in one day. | ||
| + | |style="text-align:right;"|והשני שממריקי' בשלישי' היום ממריק ג' ליום אחד | ||
| + | |- | ||
| + | | | ||
| + | ::Hence, these two pipes drain 5 wells in one day. | ||
| + | ::<math>\scriptstyle{\color{blue}{2+3=5}}</math> | ||
| + | |style="text-align:right;"|הרי ה' בורות שאילו ב' קני' ממריקי' ביום אחד | ||
| + | |- | ||
| + | | | ||
| + | ::Still, ten wells remain that the three are filling in one day, when the two are draining. | ||
| + | ::<math>\scriptstyle{\color{blue}{X=\frac{1}{15-5}=\frac{1}{10}}}</math> | ||
| + | |style="text-align:right;"|ועדיין נשארו י' בורות שהג' ממלאי' ביום אחד כשהב' מריקי‫' | ||
| + | |- | ||
| + | | | ||
| + | ::We find that the well is filled in one-tenth of a day. | ||
| + | |style="text-align:right;"|נמצא שהבור מתמלא בעשירי' היום | ||
| + | |- | ||
| + | | | ||
| + | :By this you will be able to understand everything like that and so on. | ||
| + | |style="text-align:right;"|ובזה תוכל להבין כל כזה וכיוצא בזה | ||
| + | |- | ||
| + | !<span style=color:Green>Barrel</span> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:barrel|625|8j1v}}8) For instance, if one asks: there is a barrel with three taps. When flowing from it through one tap, it is drained in one day. Through the second tap, it is drained in a half of a day. Through the third tap, it [is drained] in a third of a day. If all of the [taps] are dripping together, how long will it take the barrel to be drained? | ||
| + | :<math>\scriptstyle X+2X+3X=1</math> | ||
| + | |style="text-align:right;"|כגון <big>ח' אם</big> ישאל השואל יש כאן <sup>חבית של</sup> ג' ברזות שהוא זב ממנו דרך ברזא האחד זב היא ביום שלם ודרך ברזא השני' זב היא בחצי היום ודרך ברזא השלישי היא בשליש יום וכשהן זבין כולן כאחד בכמה החבית יוצא{{#annotend:8j1v}} | ||
| + | |- | ||
| + | | | ||
| + | ::Answer: it is drained in a sixth of a day. | ||
| + | |style="text-align:right;"|תשובה שבשישי' היום יוצא | ||
| + | |- | ||
| + | | | ||
| + | ::The tap, from which it flows in a whole day, drains one barrel in one day. | ||
| + | |style="text-align:right;"|היאך הברזא שהוא זב ממנו ביום שלם מריק' חבית אחת ביום אחד | ||
| + | |- | ||
| + | | | ||
| + | ::The second [tap], from which it flows in half a day, drains two barrels in one day. | ||
| + | |style="text-align:right;"|והשני שהוא זב בחצי יום מריקה ב' חביות ביום אחד | ||
| + | |- | ||
| + | | | ||
| + | ::The third [tap], from which it flows in a third of a day, drains three barrels in one day. | ||
| + | |style="text-align:right;"|והשלישי שהוא מריקה בשליש היום מריק' ג' חביות [ביום אחד‫] | ||
| + | |- | ||
| + | | | ||
| + | ::When they are drain together, they drain six barrels in one day. | ||
| + | ::<math>\scriptstyle{\color{blue}{1+2+3=6}}</math> | ||
| + | |style="text-align:right;"|ס"ה כשמריקי' יחד מריקי' ו' חביות ביום אחד | ||
| + | |- | ||
| + | | | ||
| + | ::We find that it is drained in a sixth of a day | ||
| + | ::<math>\scriptstyle{\color{blue}{X=\frac{1}{6}}}</math> | ||
| + | |style="text-align:right;"|נמצא שמתרוקן בשישית היום | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:barrel|625|xLF2}}9) Question: If one asks: In a barrel there are three taps. When one is dripping alone, it drains it in one hour. The second drains it in 2 hours. The third drains it in 3 hours. If all of the [taps] are dripping together, how long will it take them to drain [the barrel]? | ||
| + | :<math>\scriptstyle6X+3X+2X=12</math> | ||
| + | |style="text-align:right;"|<big>ט' אם</big> ישאל השואל הנה בחבית ג' ברזות האחד כשזב לבדו מריקו בשעה והשני לבד ממריקו בב' שעו' והשלישי מריקו בג' שעות וכשיזובו כולם כאחד בכמה מריק‫'{{#annotend:xLF2}} | ||
| + | |- | ||
| + | | | ||
| + | ::I will give you an example of a barrel containing 12 hin that is drained in half an hour plus 11 parts of half an hour. | ||
| + | |style="text-align:right;"|אבקש לך דמיונות [בחבית המחזיק]‫<ref>M: בחצי הממריק'</ref> י"ב הינין שממורק בחצי שעה ובחלק א"א שבחצי שעה | ||
| + | |- | ||
| + | | | ||
| + | ::Do as follows: | ||
| + | |style="text-align:right;"|וכה תעשה | ||
| + | |- | ||
| + | | | ||
| + | ::Say first: how much does the tap that drains in one hour drain in half an hour? 6 hin. | ||
| + | |style="text-align:right;"|אמור בתחילה הברזא שממריק בשעה כמה ממריק בחצי שעה ו' הינין | ||
| + | |- | ||
| + | | | ||
| + | ::How much does [the tap] that drains in 2 hours drain in half an hour? 3 hin. | ||
| + | |style="text-align:right;"|והממריק בב' שעות כמה ממריק בחצי שעה ג' <s>ה</s> הינין | ||
| + | |- | ||
| + | | | ||
| + | ::[The tap] that drains in 3 hours, drains 3 hin in half an hour. | ||
| + | |style="text-align:right;"|והממריק בג' שעות ממריק בחצי שעה ב' הינין | ||
| + | |- | ||
| + | | | ||
| + | ::We find that the three taps that drain in 1, 2, 3 [hours], drain 11 hin of the barrel containing 12 hin in half an hour. | ||
| + | ::<math>\scriptstyle{\color{blue}{6+3+2=11}}</math> | ||
| + | |style="text-align:right;"|נמצא שג' ברזות המריקי' א' ב' ג' מריקי' בחבית המחזיק י"ב הינין בחצי י"א היני‫' | ||
| + | |- | ||
| + | | | ||
| + | ::How much is left? One more hin. We find that this hin is drained in one part of 11 of half an hour. | ||
| + | ::<math>\scriptstyle{\color{blue}{X=\frac{12}{11}=1+\frac{1}{11}}}</math> | ||
| + | |style="text-align:right;"|כמה נשאר עוד הין נמצא שאותו הין שאותו ממריק בחלק י"א שבחצי שעה | ||
| + | |- | ||
| + | |Note well and you will understand. | ||
| + | |style="text-align:right;"|ודוק ותבין | ||
| + | |- | ||
| + | !<span style=color:Green>Fountain</span> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:four rivers|625|lOuB}}10) Another question: four rivers are flowing towards a fountain. The first fills it in a day, the second in two days, the third in 3 days, and fourth in 4 days. If all are flowing together, how long will it take [the fountain] to be filled. | ||
| + | :<math>\scriptstyle X+\frac{1}{2}X+\frac{1}{3}X+\frac{1}{4}X=1</math> | ||
| + | |style="text-align:right;"|<big>י' ועוד</big> שאלו ד' נהרות רצים אל מעיין אחד<br> | ||
| + | הראשון ממלאו ביום אחד והב' בב' והג' בג' והד' בד' ואם רצו כולם ביחד בכמה מתמלא{{#annotend:lOuB}} | ||
| + | |- | ||
| + | | | ||
| + | ::Do according to this way: | ||
| + | |style="text-align:right;"|עשה על דרך זה | ||
| + | |- | ||
| + | | | ||
| + | ::Say: where are half, a third, and a quarter are found? [by reduction as above] in 12: a half is 6, a third is 4, a quarter is 3. Sum them together; it is 25 and this is the denominator. | ||
| + | |style="text-align:right;"|אמור אחד חצי שליש רביע ‫<ref>121r</ref>אנה ימצאון [ר"ל על דרך המועט כדלעיל דהיינו] בי"ב האחד <s>ה</s> י"ב חצי ו' שליש ד' רביע ג' צרפם יחד הרי כ"ה וזה המורה | ||
| + | |- | ||
| + | | | ||
| + | ::We find that in 12 days they fill 25 fountains: | ||
| + | |style="text-align:right;"|נמצא בי"ב ימי' ימלאו כ"ה מענייות | ||
| + | |- | ||
| + | | | ||
| + | ::The first [river] fills 12 fountains in 12 days. | ||
| + | |style="text-align:right;"|כיצד הראשון ממלאו' בי"ב ימים י"ב מענייות | ||
| + | |- | ||
| + | | | ||
| + | ::The second [river fills] 6 fountains in 12 days. | ||
| + | |style="text-align:right;"|השני בי"ב ימי' ו' מעיינות | ||
| + | |- | ||
| + | | | ||
| + | ::The third [river fills] 4 fountains in 12 days. | ||
| + | |style="text-align:right;"|השלישי בי"ב ימי' ד' מעיינות | ||
| + | |- | ||
| + | | | ||
| + | ::The fourth [river fills] 3 fountains in 12 days. | ||
| + | |style="text-align:right;"|הרביעי' בי"ב ימי' ג' מעיינות | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | ::<math>\scriptstyle{\color{blue}{12+\left(\frac{1}{2}\sdot12\right)+\left(\frac{1}{3}\sdot12\right)+\left(\frac{1}{4}\sdot12\right)=12+6+4+3=25}}</math> | ||
| + | |- | ||
| + | | | ||
| + | ::<span style=color:Green>'''Rule of Three:'''</span> so, [if] 25 fountains [are filled] in 12 days, how long will it take [to fill] one fountain? | ||
| + | |style="text-align:right;"|א"כ כ"ה מעיינות בי"ב ימי' מעיין אחד בכמה | ||
| + | |- | ||
| + | | | ||
| + | ::Then, multiply 1 by 12; the result is 12. Divide it [by 25], according to the chapter of division, [meaning how many times 25 is in 12]; we find that one fountain is filled in 12 parts of which 25 is a whole day. | ||
| + | ::<math>\scriptstyle{\color{blue}{X=\frac{1\sdot12}{25}=\frac{12}{25}}}</math> | ||
| + | |style="text-align:right;"|אז כפול א' בי"ב ויעלה י"ב חלקי' בשער החילוק [בכ"ה פי' כמה פעמים כ"ה יש בי"ב כנ"ל אש'] נמצא שמעיין האחד מתמלא בי"ב חלקי' שכ"ה מהן יום שלם | ||
| + | |- | ||
| + | | | ||
| + | ::To know how much water flows through the first river: multiply 12 by 1; the result is 12. Divide it by 25, [according to the chapter of division]; we find that the water is 12 parts of 25. | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{1\sdot12}{25}=\frac{12}{25}}}</math> | ||
| + | |style="text-align:right;"|לידע כמה חלק מים של כל נהרות לראשון כפול י"ב בא' ויעלה י"ב חלקי' [בשער החילוק] בכ"ה נמצא שמימיו י"ב חלקי' מכ"ה | ||
| + | |- | ||
| + | | | ||
| + | ::For the second [rever]: multiply 6 by 1; the result is 6. Divide it by 25, [according to the chapter of division]; it is 6 parts of 25. | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{1\sdot6}{25}=\frac{6}{25}}}</math> | ||
| + | |style="text-align:right;"|כפול ו' לשנים בא' ויעלה ו' חלקי' [בשער החילוק] בכ"ה הרי ו' חלקי' מכ"ה | ||
| + | |- | ||
| + | | | ||
| + | ::For the third [river]: multiply 4 by 1. | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{1\sdot4}{25}}}</math> | ||
| + | |style="text-align:right;"|לשלישי כפול ד' בא‫' | ||
| + | |- | ||
| + | | | ||
| + | ::For the fourth [river]: [multiply] 3 by 1. | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{1\sdot3}{25}}}</math> | ||
| + | |style="text-align:right;"|לרביעי ג' בא‫' | ||
| + | |- | ||
| + | | | ||
| + | :Note well and you will understand. | ||
| + | |style="text-align:right;"|ודוק ותבין | ||
| + | |- | ||
| + | ! | ||
| + | |||
| + | ==== <span style=color:Green>Multiple Quantities Problems - Selling Eggs</span> ==== | ||
| + | |||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:selling eggs|652|CQiB}}11) Pose a riddle: three women are selling eggs. One has 10 eggs, the second has 30, and the third has 50. Each of them gives a certain amount of eggs for one ḥalish and they earns the same amount of money | ||
| + | :<math>\scriptstyle10X=30Y=50Z</math> | ||
| + | |style="text-align:right;"|<big>י"א חוד</big> חידה ג' נשי' מוכרות ביצי' לאחת יש י' ביצים לשנית יש ל' ולשלישי' נ' וכל אחת תתן ביצה בחליש אחד כמו חבירת' ויפדו מעות שוות{{#annotend:CQiB}} | ||
| + | |- | ||
| + | | | ||
| + | ::Answer: each one will give 7 [eggs] for one ḥalish and what remains on the seventh - she will give one egg for 3 ḥalish | ||
| + | |style="text-align:right;"|<big>תשובה</big> כל אחת תתן ז' בחליש וכל מה שנותר על השביעיות תתן ביצה אחת בעד ג' חלישים | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{OliveGreen}{\begin{cases}\scriptstyle1+\left[3\sdot\left[10-\left(1\sdot7\right)\right]\right]=1+\left[3\sdot\left(10-7\right)\right]=1+\left(3\sdot3\right)\\\scriptstyle4+\left[3\sdot\left[30-\left(4\sdot7\right)\right]\right]=4+\left[3\sdot\left(30-28\right)\right]=4+\left(3\sdot2\right)\\\scriptstyle7+\left[3\sdot\left[50-\left(7\sdot7\right)\right]\right]=7+\left[3\sdot\left(50-49\right)\right]=7+\left(3\sdot1\right)\end{cases}}}</math> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :*If they are four [women]. The fourth has 70 - the calculation is the same. | ||
| + | |style="text-align:right;"|ואם היית' ארבע לרביעי' לה ע' ביצים והחשבון כמו כן | ||
| + | |- | ||
| + | ! | ||
| + | |||
| + | ==== <span style=color:Green>Proportional Division - Inheritance</span> ==== | ||
| + | |||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:inheritance|645|8Dgv}}12) Question: an inheritance was given to three brothers. | ||
| + | :The first took as much as he wanted, and so did the second and the third. | ||
| + | :Then the second and the third returned to first and complained against him: you took more than you deserved, therefore you should share with us equally. | ||
| + | :He answered: do not judge me, I will give each of you the same amount as he has, be it is much or less; and so he did. | ||
| + | :Later the first and the third returned to the second and he answered them the same as the first. | ||
| + | :Afterwards the first and the second returned to the third and he too said the same as the previous. | ||
| + | :Now the division between them became equal. | ||
| + | :How much did each of them took at the beginning and how much is the whole amount of the money? | ||
| + | |style="text-align:right;"|<big>י"ב שאלה</big> אם נפלה ירושה לפני ג' אחים<br> | ||
| + | הראשון לקח כל מה שירצה וכן השני וכן השלישי<br> | ||
| + | ואח"כ חוזרים השני והשלישי על הראשון וטוענים אותו נטלת מן הראוי לך לכן תחלק עמנו שוה בשוה<br> | ||
| + | והוא אמר אל תדונו עמי אתן לכל אחד מכם כמו שיש לו כבר הן רב הן מעט וכן עשה<br> | ||
| + | ואח"כ חוזר הראשון והשלישי על השני והוא משיב ג"כ להם כמו הראשון<br> | ||
| + | ואח"כ חזרו הראשון והשני על השלישי והוא אומר ג"כ כמו הראשוני‫'<br> | ||
| + | ואז יהיה החולקה שוה ביניהם<br> | ||
| + | חוד כמה לקח כל אחד בתחילה או כמה סך המעות ביחד{{#annotend:8Dgv}} | ||
| + | |- | ||
| + | | | ||
| + | ::Answer: the total is 24; the first took 13; the second 7; and the third 4. | ||
| + | ::<math>\scriptstyle{\color{blue}{a_1+a_2+a_3=24}}</math> | ||
| + | |style="text-align:right;"|<big>תשובה</big> הכל היה כ"ד הראשו' לקח י"ג והשני ז' והשלישי ד‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Here is the instruction of the problem: | ||
| + | |style="text-align:right;"|והילך המורה את החידה | ||
| + | |- | ||
| + | | | ||
| + | ::If they are only three, take for the last 3 plus one more; it is 4. | ||
| + | ::<math>\scriptstyle{\color{blue}{a_3=3+1=4}}</math> | ||
| + | |style="text-align:right;"|אם הם רק ג' אז קח לאחרון ג' ואחד יותר הרי זהו ד‫' | ||
| + | |- | ||
| + | | | ||
| + | ::For the middle take twice as much as the last, which is 8, but subtract one; it is 7. | ||
| + | ::<math>\scriptstyle{\color{blue}{a_2=\left(2\sdot a_3\right)-1=\left(2\sdot4\right)-1=8-1=7}}</math> | ||
| + | |style="text-align:right;"|ולאמצעי קח כפלים כמו לאחרון וזהו ח' רק אחת תסיר ויהיה ז‫' | ||
| + | |- | ||
| + | | | ||
| + | ::For the first take twice as much as the middle, which is 14, and subtract one; it is 13. | ||
| + | ::<math>\scriptstyle{\color{blue}{a_1=\left(2\sdot a_2\right)-1=\left(2\sdot7\right)-1=14-1=13}}</math> | ||
| + | |style="text-align:right;"|ולראשון קח כפלים כמו לאמצעי דהיינו י"ד רק אחת תסיר <s>ויהו</s> ויהיה י"ג | ||
| + | |- | ||
| + | | | ||
| + | :And so on, as many as they are, take for the last the number they are plus one more, then double the required and subtract 1. | ||
| + | |style="text-align:right;"|וכן לעולם כמה הן אז קח לאחרון כמניין כולם וא' יותר ואח"כ כפול המבוקש ותסיר אחת | ||
| + | |- | ||
| + | | | ||
| + | :*If they were four [brothers]. | ||
| + | |style="text-align:right;"|וכן אם הם ד‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Take for the last 4 plus one more; it is 5. | ||
| + | ::<math>\scriptstyle{\color{blue}{a_4=4+1=5}}</math> | ||
| + | |style="text-align:right;"|קח לאחרון ד' וא' יותר וזהו ה‫' | ||
| + | |- | ||
| + | | | ||
| + | ::For the third ten and subtract one; it is 9. | ||
| + | ::<math>\scriptstyle{\color{blue}{a_3=\left(2\sdot a_4\right)-1=\left(2\sdot5\right)-1=10-1=9}}</math> | ||
| + | |style="text-align:right;"|ולשלישי י' ואחד תסיר זהו ט‫' | ||
| + | |- | ||
| + | | | ||
| + | ::For the second 18 and subtract one; it is 17. | ||
| + | ::<math>\scriptstyle{\color{blue}{a_2=\left(2\sdot a_3\right)-1=\left(2\sdot9\right)-1=18-1=17}}</math> | ||
| + | |style="text-align:right;"|לשני י"ח ואחד תסיר זהו י"ז | ||
| + | |- | ||
| + | | | ||
| + | ::For the first 34 and subtract one; it is 33. | ||
| + | ::<math>\scriptstyle{\color{blue}{a_1=\left(2\sdot a_2\right)-1=\left(2\sdot17\right)-1=34-1=33}}</math> | ||
| + | |style="text-align:right;"|לראשון ל"ד ואחד תסיר זהו ל"ג | ||
| + | |- | ||
| + | | | ||
| + | :''Consider well him that is before thee'' [Proverbs 23, 1]. | ||
| + | |style="text-align:right;"|''{{#annot:Pr23-1|494|ueH4}}בין תבין את אשר לפניך{{#annotend:ueH4}}''‫<ref group=note>משלי כג, א</ref> | ||
| + | |- | ||
| + | ! | ||
| + | |||
| + | ==== <span style=color:Green>Payment Problem - Digging a Hole</span> ==== | ||
| + | |||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :{{#annot:digging a hole|612|aTjq}}13) A worker was hired to dig a hole with a length of 17 cubits and a width of 7 cubits for 33 zehuvim, but the worker dug [a hole with a width of] 3 cubits and a length of 17 cubits. How much should be his payment? | ||
| + | :<math>\scriptstyle\frac{7\sdot17}{33}=\frac{3\sdot17}{X}</math> | ||
| + | |style="text-align:right;"|‫<ref>121v</ref><big>יג</big>‫<ref>Paris 1088 יט</ref> <big>שוכר</big> את הפועל לחפור בור ארוכה י"ז אמות ברוחב ז' אמות בעד ל"ג זהו' והפועל חפר ג' אמו' במשך י"ז<br> | ||
| + | כמה שכרו{{#annotend:aTjq}} | ||
| + | |||
| + | |- | ||
| + | | | ||
| + | ::Answer: first, multiply 7 times 17 according to the chapter on multiplication; the result is 119. | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{7\sdot17}{119}}}</math> | ||
| + | |style="text-align:right;"|תשובה כפול בתחילה בשער הכפל ז"פ י"ז ויעלה טא"א | ||
| + | |- | ||
| + | | | ||
| + | ::We find that he hires the worker to dig 119 cubits breadthwise for 33 zehuvim. | ||
| + | |style="text-align:right;"|נמצא ששכר את הפועל לחפור קי"ט אמו' ברוחב אמה בעד ל"ג זהו‫' | ||
| + | |- | ||
| + | | | ||
| + | ::The worker digs 3 cubits lengthwise. So, multiply 3 by 17; the result is 51. | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{3\sdot17}{51}}}</math> | ||
| + | |style="text-align:right;"|והפועל חפר ג' אמות במשך י"ז כפול ג' בי"ז ויעלה א"ה | ||
| + | |- | ||
| + | | | ||
| + | ::We find that he digs only 51 cubits, but he has to dig 119 cubits. | ||
| + | |style="text-align:right;"|נמצא שלא חפר רק נ"א אמות והיה לו לחפור קי"ט אמות | ||
| + | |- | ||
| + | | | ||
| + | ::Then, write 119 cubits and beneath it write 51, like this: | ||
| + | ::<math>\scriptstyle\frac{119}{33}=\frac{51}{X}</math> | ||
| + | |style="text-align:right;"|ואח"כ כתוב קי"ט אמו' ולמטה הימנו כתוב נ"א אמו' כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color: blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |1||1||9 | ||
| + | |- | ||
| + | | ||3||3 | ||
| + | |- | ||
| + | | ||5||1 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |א||א||ט | ||
| + | |- | ||
| + | | ||ג||ג | ||
| + | |- | ||
| + | | ||ה||א | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::<span style=color:Green>'''Rule of Three:'''</span> multiply 51 by 33 according to the chapter on multiplication; the result is [1]683. | ||
| + | |style="text-align:right;"|וכפול בשער הכפל א"ה על ג'ג' ויעלה תרפ"ג | ||
| + | |- | ||
| + | | | ||
| + | ::Divide 1683 by 119, meaning how many times 119 is in [1]683; you find 14 times 119 and 17 parts, of which 119 is a whole. | ||
| + | ::<math>\scriptstyle{\color{blue}{X=\frac{33\sdot51}{119}=\frac{1683}{119}=14+\frac{17}{119}}}</math> | ||
| + | |style="text-align:right;"|‫[חלוק ג"חו"א] לקי"ט פי' כמה פעמי' קי"ט איתא בתרפ"ג ותמצא י"ד פעמי' קי"ט דהיינו טא"א וי"ז חלקי' שלא הגיעו לקי"ט שהוא חלק שלם | ||
| + | |- | ||
| + | | | ||
| + | ::We find that the payment of the worker is 14 zehuvim and 17 parts, of which 119 is a whole zahuv. | ||
| + | |style="text-align:right;"|נמצא שכר הפועל י"ד זהו' וי"ז החלקי' שקי"ט מהן חלק זהוב שלם | ||
| + | |- | ||
| + | ! | ||
| + | |||
| + | ==== <span style=color:Green>Find a Quantity Problem - Stolen Purse</span> ==== | ||
| + | |||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :{{#annot:stolen purse|628|vtCy}}14) A man gave his purse with some money to his friend for safekeeping and it was stolen. Both the depositor and the keeper do not know how much money was in the purse. The depositor remembers that when he counted the money by two 1 remained, by three 1 remained, by four 1 remained, by five 1 remained, by six 1 remained, and by seven nothing remained. | ||
| + | :<math>\scriptstyle2a_1+1=3a_2+1=4a_3+1=5a_4+1=6a_5+1=7a_6</math> | ||
| + | |style="text-align:right;"|<big>יד אדם</big> הפקיד לחבירו כיסו מלא מעות ונגנב בפשיעה והמפקיד והנפקד אינם יודעי' כמה היו המעות אשר בו אלא המפקיד זוכר כשמנה המעות בב"ב נשאר א' בג"ג נשאר א' בד'ד' נשאר א' בה"ה נשאר א' בו'ו' נשאר א' בז' יצא מכוון [והנה חוד כמה היו המעות הללו]{{#annotend:vtCy}} | ||
| + | |- | ||
| + | | | ||
| + | ::Answer: the coins are 301 or 721. | ||
| + | |style="text-align:right;"|תשובה <s>מ</s> המעות ההם א' וג' מאות וסימנך וא"ש יצא מחשבון או תשכ"א היו המעט | ||
| + | |- | ||
| + | | | ||
| + | ::I will teach you how to solve it: | ||
| + | |style="text-align:right;"|והנה אשכילך הדרך היאך תעשה | ||
| + | |- | ||
| + | | | ||
| + | ::For every number in the question write the least you find, meaning: you ask for a number that is counted by 2 and 1 remains - write 2 for it; you ask for a number counted by 3 - write 3 for it; you ask for a number counted by 4 - write 4 for it; you ask for a number counted by 5 - write 5; you ask for a number counted by 6 - write 6. | ||
| + | |style="text-align:right;"|כתוב כנגד כל מניין השאלה מספר המעוט שתצא בו השאלה פי' שאלת מניין הנמנה בב'ב' וישאר א' נגד [.] זה כתוב ב' ושאלת מניין הנמנה ג'ג' נגד זה כתוב ג' וששאלת מניין הנמנה ד'ד' נגד זה כתוב ד' וששאלת מניין הנמנה ה'ה' נגד זה כתוב ה' ושאלת מנין הנמנה ו'ו' נגד זה כתוב ו‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Then, multiply 2 by 3; the result is 6. | ||
| + | |style="text-align:right;"|ואח"כ כפול ב' על ג' ויעלה ו‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Multiply 6 by 4; the result is 24. | ||
| + | |style="text-align:right;"|כפול ו' על ד' ויעלה ד'ב‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Multiply 24 by 5; the result is 120. | ||
| + | |style="text-align:right;"|כפול ד'ב' על ה' ויעלה 0ב"א | ||
| + | |- | ||
| + | | | ||
| + | ::Multiply 120 by 6; the result is 720, meaning 7 hundred and twenty and this is a number divisible by 2, 3, 4, 5, and 6. | ||
| + | |style="text-align:right;"|כפול 0ב"א על ו' ויעלה 0ב"ז פי' עשרי' וז' מאות זהו מספר המתחלק לב' ולג' ולד' ולה' ולו‫' | ||
| + | |- | ||
| + | | | ||
| + | ::You add to it one more, so it becomes 721, and when you divide it by 2, 3, 4, 5, or 6, the remainder is 1 for each [of these] numbers; divide it by 7 and [there is no remainder]. | ||
| + | |style="text-align:right;"|ובהוסיפך עוד אחד שיהיה א"ב אז כשתחלקהו לבגדה"ו ישאר בכל המניין אחת וכשתחלקהו לז'ז' יצא [.] מכוון | ||
| + | |- | ||
| + | |colspan="2"| | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(2\sdot3\sdot4\sdot5\sdot6\right)+1=\left(6\sdot4\sdot5\sdot6\right)+1=\left(24\sdot5\sdot6\right)+1=\left(120\sdot6\right)+1=720+1=721}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :*If the number is divided by 2, 3, 4, 5, 6, 7, 8 so that the remainder is 1 and by 9 [there is no remainder]. | ||
| + | |style="text-align:right;"|וכן אם ישאל השואל מספר המתחלק לבגדהוז"ח וישאר א' ובט' יצא מכוון | ||
| + | |- | ||
| + | |colspan="2"| | ||
| + | ::<math>\scriptstyle2a_1+1=3a_2+1=4a_3+1=5a_4+1=6a_5+1=7a_6+1=8a_7+1=9a_8</math> | ||
| + | |- | ||
| + | | | ||
| + | ::Multiply 7 by 8; the result is 56. | ||
| + | |style="text-align:right;"|אזי כפול ז"ח ויעלה ו"ה | ||
| + | |||
| + | |- | ||
| + | | | ||
| + | ::Multiply 56 by 720; the result is divisible by 2, 3, 4, 5, 6, 7, and 8. | ||
| + | |style="text-align:right;"|ואח"כ כפול ו"ה על 0ב"ז והעולה הוא המתחלק לבגדהוז"ח | ||
| + | |- | ||
| + | | | ||
| + | ::When you add one to it, 1 remains for each [of these] numbers and by 9 [there is no remainder]. | ||
| + | ::<math>\scriptstyle{\color{blue}{\left[720\sdot\left(7\sdot8\right)\right]+1=\left(720\sdot56\right)+1}}</math> | ||
| + | |style="text-align:right;"|‫<ref>122r</ref>ובהוסיפך אחת עליו ישאר א' בכל המניין וב' יוצא מכוון | ||
| + | |- | ||
| + | | | ||
| + | :*If one asks for a number, such that the remainder from all the numbers [2-6] is 3, add 3 to the product after you multiply all [these] numbers. | ||
| + | ::<math>\scriptstyle2a_1+3=3a_2+3=4a_3+3=5a_4+3=6a_5+3=7a_6</math> | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(2\sdot3\sdot4\sdot5\sdot6\right)+3}}</math> | ||
| + | |style="text-align:right;"|ואם ישאלך מניין הנותרי' ג' בכל המנייני' אזי תוסיף ג' על העולה אחר כופלך מספרי' המועטי‫' | ||
| + | |- | ||
| + | | | ||
| + | :*Likewise, if you are asked [for a number], such that the remainder is 4, add 4 and so on this way. | ||
| + | ::<math>\scriptstyle2a_1+4=3a_2+4=4a_3+4=5a_4+4=6a_5+4=7a_6</math> | ||
| + | ::<math>\scriptstyle{\color{OliveGreen}{\left(2\sdot3\sdot4\sdot5\sdot6\right)+4}}</math> | ||
| + | |style="text-align:right;"|וכן אם ישאלך [מניין הנותר] ד' אזי תוסיף ד' וכן לעולם | ||
| + | |- | ||
| + | ! | ||
| + | |||
| + | ==== <span style=color:Green>Find a Quantity Problem - How Much Problem - Amount of Money</span> ==== | ||
| + | |||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:money|648|pRTq}}15) Question: we add to an amount of money its half, its third, its quarter, its fifth, and its sixth, and the total is 40. How much was the original amount of money? | ||
| + | :<math>\scriptstyle X+\frac{1}{2}X+\frac{1}{3}X+\frac{1}{4}X+\frac{1}{5}X+\frac{1}{6}X=40</math> | ||
| + | |style="text-align:right;"|<big>ט"ו שאלה</big> ממון הוספנו עליו מחציתו שלישיתו רביעיתו חמישיתו שישיתו והכל הוא מ‫'<br> | ||
| + | כמה היה הממון{{#annotend:pRTq}} | ||
| + | |- | ||
| + | | | ||
| + | ::First, I will tell you what is in my heart: take the least number divisible into halves, thirds, quarters, fifths, and sixths; it is 60, meaning sixty. | ||
| + | |style="text-align:right;"|בתחילה אשכילך את אשר בלבבי קח מספר המועט המתחלק לחצאין לשליש ולרביע ולחומשי' ולששין וזהו 0"ו פי' ששים | ||
| + | |- | ||
| + | | | ||
| + | ::Add all the parts you are asked for to sixty: the half, which is 30; the third, which is twenty; the quarter, which is 15; the fifth, [which is] 12; and the sixth, [which is] 10. Sum them together; it is 87. Add 87 to sixty; the result is 147. | ||
| + | |style="text-align:right;"|ותוסיף על ששים כל החלקי' ששאלת החצי הוא ל' והשליש הוא עשרי' והרביעי הוא ט"ו והחמשי' י"ב והששית י' וצרפם הכל יחד ויהיה פ"ז חבור פ"ז עם ששים ויעלה קמ"ז | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | ::<math>\scriptstyle{\color{blue}{\begin{align}&\scriptstyle60+\left(\frac{1}{2}\sdot60\right)+\left(\frac{1}{3}\sdot60\right)+\left(\frac{1}{4}\sdot60\right)+\left(\frac{1}{5}\sdot60\right)+\left(\frac{1}{6}\sdot60\right)\\&\scriptstyle=60+30+20+15+12+10=60+87=147\\\end{align}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | ::<span style=color:Green>'''Rule of Three:'''</span> We find that if you were asked for an amount of money, such that you add to it its half, its third, its quarter, its fifth, and its sixth, and the total is 147, how much is the original amount of money? We would answer that the original amount is sixty. Now that it is only 1, how much is the original amount? | ||
| + | ::<math>\scriptstyle{\color{blue}{147:60=40:X}}</math> | ||
| + | |style="text-align:right;"|נמצא אם היו שואלי' אותך ממון שהוספת עליו מחציתו שלישיתו ורביעיתו חמשיתו ששיתו ובין הכל קמ"ז כמה תחילת הממון היינו משיבי' תחילתו ששים ועתה שהוא בין הכל רק א' כמה היה תחילתו | ||
| + | |- | ||
| + | | | ||
| + | ::Write 147 on the top line, a little far from it write the sixty, and below the 60 write the 40 we are asking for, like this: | ||
| + | |style="text-align:right;"|אז כתוב אותן קמ"ז בטור העליון ורחוק ממנו קצת כתוב הששים ותחת הס' כתוב המ' שאנו שואלי' ויהיה כך | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color: blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |60||147 | ||
| + | |- | ||
| + | |40|| | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |‫0ו||זדא | ||
| + | |- | ||
| + | |‫0ד|| | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Multiply 40 by 60 above it; the result is 2400, meaning 24 hundreds. | ||
| + | |style="text-align:right;"|כפול 0"ד ב 0"ו שעליהם ויעלה 00ד"ב פי' כ"ד מאות | ||
| + | |- | ||
| + | | | ||
| + | ::See how many times 147 is in 24 hundreds; you find that there are 16 times 147 in it and 48 remain that do not add up to 147. | ||
| + | ::<math>\scriptstyle{\color{blue}{X=\frac{40\sdot60}{147}=\frac{2400}{147}=16+\frac{48}{147}}}</math> | ||
| + | |style="text-align:right;"|תחלק כמה פעמי' זד"א בכ"ד מאות ותמצא שיש בו י"ו פעמי' זד"א ונותר מ"ח חלקי' (חלקי') קטני' שאינן מגיעי' לזד"א | ||
| + | |- | ||
| + | | | ||
| + | ::We find that the original amount of money was 16 integers and 48 parts, of which 147 is a whole unit. | ||
| + | |style="text-align:right;"|נמצא שתחילת הממון י"ו שלמי' ומ"ח חלקי' שזד"א עושי' חלק שלם | ||
| + | |- | ||
| + | | | ||
| + | ::<span style=color:Green>'''Check:'''</span> if you want to check if your calculation is correct, multiply 147 by 16, meaning how much is 16 times 147; the result is 2352, meaning 2 thousand, 3 hundred and 52. | ||
| + | |style="text-align:right;"|ואם תחפוץ לשקול אם חשבונך מכוון אז כפול זד"א על י"ו פי' כמה י"ו פעמי' זד"א ויעלה בהג"ב פי' ב' אלפי' וג' מאות ונ"ב | ||
| + | |- | ||
| + | | | ||
| + | ::Add to it the 48 remaining above that is less than 147; it is 2400, meaning 24 hundreds. | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(147\sdot16\right)+48=2352+48=2400}}</math> | ||
| + | |style="text-align:right;"|חבר עמהם מ"ח הנותרי' למעלה שלא עלו לזד"א ויהיה 00ד"ב פי' כ"ד מאות | ||
| + | |- | ||
| + | | | ||
| + | ::Add to it its half, its third, its quarter, its fifth, and its sixth. Sum all with the 24 hundred. | ||
| + | |style="text-align:right;"|תוסיף עליהם החציה השלישי' והרביעי' החמישי' והששי' וחבור הכל עם הכ"ד מאות | ||
| + | |- | ||
| + | |colspan="2"| | ||
| + | ::<math>\scriptstyle{\color{blue}{2400+\left(\frac{1}{2}\sdot2400\right)+\left(\frac{1}{3}\sdot2400\right)+\left(\frac{1}{4}\sdot2400\right)+\left(\frac{1}{5}\sdot2400\right)+\left(\frac{1}{6}\sdot2400\right)}}</math> | ||
| + | |- | ||
| + | | | ||
| + | ::Then, multiply 40, meaning 40 times, by 147. | ||
| + | ::<math>\scriptstyle{\color{blue}{40\sdot147}}</math> | ||
| + | |style="text-align:right;"|ואח"כ כפול 0"ד פי' מ' פעמי' בזד"א | ||
| + | |- | ||
| + | | | ||
| + | ::If you find that what you get after you multiply 40 by 147 is the same as the number you find when you add half, third, quarter, fifth, and sixth to 24 hundreds, then your calculation is correct, otherwise you are wrong. | ||
| + | |style="text-align:right;"|ואם תמצא מכוון מה שיעלה לך אחר שתכפול מ' בקמ"ז כמו החשבון שמצאת ‫<ref>122v</ref>בהוסיפך אבגדה"ו על כ"ד מאות אז חשבונך מכוון ואם לאו ודאי | ||
| + | טעית | ||
| + | |- | ||
| + | | | ||
| + | :The one who [understands] will understand. | ||
| + | |style="text-align:right;"|והבמין יבין | ||
| + | |- | ||
| + | ! | ||
| + | |||
| + | ==== <span style=color:Green>Divide a Quantity - Loans Repayment</span> ==== | ||
| + | |||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:loans repayment|644|7djb}}16) If you are asked: Reuven and Shimon lent two loans: | ||
| + | :Reuven lent 37 zehuvim with an interest of one pašuṭ for each zahuv every week for 55 weeks. | ||
| + | :Shimon lent to the same borrower 23 zehuvim for 33 weeks. | ||
| + | :Then, came the borrower and said to them: I will give both of you [1]3 zehuvim together and you will compromise. | ||
| + | :<math>\scriptstyle\frac{1}{37\sdot55}X+\frac{1}{23\sdot33}X=13</math> | ||
| + | |style="text-align:right;"|<big>י"ו אם</big> ישאלך השואל הנה ראובן שמעון הלוי' שני חובות ראובן הלויה ל"ז זהו' ועלה על כל זהב פשי' לשבוע ועמדו נ"ה שבועו' ושמעו' הלוה לאותו חייב כ"ג זהו' ועמדו ל"ג זהו' ואח"כ בא הבעל חוב ואמ' להן אתן לכם ג' זהו' מן הכל ואתם התפשרו יחד{{#annotend:7djb}} | ||
| + | |- | ||
| + | | | ||
| + | ::I will teach you the way by which you find how much should Reuven receive and how much should Shimon receive from the 13 zehuvim of the interest. | ||
| + | |style="text-align:right;"|ואשכילך הדרך אשר תמצא בו כמה מגיע לראובן וכמה מגיע לשמעון מן הי"ג זהו' כאשר קבלתי מרבי‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Multiply Reuven's interest, which is 37, by 55; the result is 2035 pešiṭim, meaning 2 thousand and 35 and this is the interest due to Reuven by law. | ||
| + | ::<math>\scriptstyle{\color{blue}{37\sdot55=2035}}</math> | ||
| + | |style="text-align:right;"|חשבון כמה רבותו של ראובן שהוא ל"ז על נ"ה ויעלה הג0"ב פשי' פי' ב' אלפי' ול"ה זה היה ריבית שהיה שייך מדינה לראובן | ||
| + | |- | ||
| + | | | ||
| + | ::Then, multiply Shimon's 23 zehuvim by 33 weeks; the result is 759 due to Shimon. | ||
| + | ::<math>\scriptstyle{\color{blue}{23\sdot33=759}}</math> | ||
| + | |style="text-align:right;"|ואח"כ כפול הכ"ג זהו' של שמעון בל"ג שבועות ויעלה טהג"ז שהיה ראוי לשמעון | ||
| + | |- | ||
| + | | | ||
| + | ::Add 759 to 2035; the result is 2[7]94 and this is the denominator. | ||
| + | ::<math>\scriptstyle{\color{blue}{2035+759=2794}}</math> | ||
| + | |style="text-align:right;"|ואח"כ חבר טה"ז עם הג0"ב ויעלה דט0"ב וזהו המורה את החידה | ||
| + | |- | ||
| + | | | ||
| + | ::Multiply the 13 zehuvim by 2035, which is the interest due to Reuven; the result is 26455. | ||
| + | |style="text-align:right;"|אח"כ כפול הי"ג זהו' על הג0"ב שהיה רבי' שהיה שייך <s>לשמעון</s> לראובן ויעלה ההדו"ב | ||
| + | |- | ||
| + | | | ||
| + | ::Divide it by the denominator, which is 2794, meaning how many times 2794 is in 26455; you find it is 9 times in it and 1309 remains that is less than 2794. | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{13\sdot2035}{2794}=\frac{26455}{2794}=9+\frac{1309}{2794}}}</math> | ||
| + | |style="text-align:right;"|אח"כ נכהו מן המורה שהוא דטז"ב פי' כמה פעמי' דטז"ב ב' ההדו"ב ותמצא שיש בו ט' פעמי' ונותרי' ט' וג' מאות ואלף שלא יעלו למניין דטז"ב | ||
| + | |- | ||
| + | | | ||
| + | ::We find that Reuven's share of the 13 zehuvim is 9 zehuvim and 1309 parts that are less than 2794. | ||
| + | |style="text-align:right;"|נמצא של ראובן שייך מן הי"ג זהו' ט' זהו' ואלף וש"ט חלקי' שלא הגיעו לכלל דטז"ב | ||
| + | |- | ||
| + | | | ||
| + | ::If you want to know how much is due to Shimon by the same way we used regarding to Reuven, multiply 13 by 759, which is Shimon's interest; the result is 9867. | ||
| + | |style="text-align:right;"|ואם תרצה לידע כמה מגיע לשמעון על הדרך שאמרנו על ראובן אז כפול י"ג בטה"ז שהוא רביתו של שמעון ויעלה זוח"ט | ||
| + | |- | ||
| + | | | ||
| + | ::Divide it by the denominator, which is 2794, meaning how many times 2794 is in 9867; you find it is 3 times in it and 1485 parts remain, of which 2794 is a whole unit, i.e. one zahuv. | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{13\sdot759}{2794}=\frac{9867}{2794}=3+\frac{1485}{2794}}}</math> | ||
| + | |style="text-align:right;"|נכיהו מן המורה שהוא דטז"ב פי' כמה פעמי' דטז"ב בזוח"ט ותמצא שיש בו ג' פעמי' ונותרי' אלף ותפ"ה חלקי' שדטז"ב מהן עושין חלק שלם דהיינו זהב | ||
| + | |- | ||
| + | | | ||
| + | ::We find that Shimon's share is 3 zehuvim and 1485 parts, of which 2794 is one zahuv. | ||
| + | |style="text-align:right;"|נמצא ששייך לשמעון ג' זהו' והחד"א חלקי' שדטז"ב מהן זהב שלם | ||
| + | |- | ||
| + | | | ||
| + | ::<span style=color:Green>'''Check:'''</span> Add 1485 to Reuven's remaining 1309; the result is 2794. | ||
| + | ::<math>\scriptstyle{\color{blue}{1309+1485=2794}}</math> | ||
| + | |style="text-align:right;"|חבר החד"א עם אלף וש"ט הנותרי' בראובן ויעלה דטז"ב | ||
| + | |- | ||
| + | | | ||
| + | ::If you want to know the number more closely divide the 13 zehuvim into pešiṭim, then multiply them by the 26455 of Reuven's interest, or by 2794 of Shimon's interest, so 2794 is divided into parts of a pašuṭ. | ||
| + | |style="text-align:right;"|ואם תחפוץ לידע חשבון בצימצום אז חלק הי"ג זהו' לפשיטי' ‫<ref>123r</ref>ואח"כ כפול בהן ההדו"ב ריביתו של ראובן או דטז"ב רביתו של שמעון ואז יתחלק דטז"ב לחלקי' פשוט‫' | ||
| + | |- | ||
| + | | | ||
| + | :Note well. | ||
| + | |style="text-align:right;"|ודוק | ||
| + | |- | ||
| + | ! | ||
| + | |||
| + | ==== <span style=color:Green>Multiple Quantities - Weights</span> ==== | ||
| + | |||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:four weights|652|r0NH}}17) If one asks you how do you find four weights weighing together 40, or 5 weights weighing together 121, so that you can weigh with them the heavy as well as the light up to 40. How much will each of them weigh by itself? | ||
| + | |style="text-align:right;"|<big>י"ז אם</big> ישאלך אדם איך תמצא ארבע משקולות שמשקל כולן רק ארבעי' ואת' תוכל לשקול בהן הן רב הן מעט עד ארבעי' כמה משקל כל אחד בפני עצמו<br> | ||
| + | או אם ה' משקל שמשקלם קכ"א ואתה תוכל לשקול בהן הן רב הן מעט כמה משקל כל אחד בפני עצמו{{#annotend:r0NH}} | ||
| + | |- | ||
| + | | | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle\sum_{i=1}^4 a_i =40\ \quad \sum_{i=1}^5 a_i =121\\\scriptstyle a_i=3^{i-1}\\\scriptstyle a_i:a_{i+1}=a_{i+1}:a_{i+2}\end{cases}</math> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | ::Do as follows: | ||
| + | |style="text-align:right;"|כה תעשה | ||
| + | |- | ||
| + | | | ||
| + | ::If you want to sum up weights: the first weighs one liṭra; the second weighs 3 liṭra; the third weighs 9 liṭra, the fifth 81 liṭra; the fourth 27 liṭra. | ||
| + | |style="text-align:right;"|אם תחפוץ להוסיף משקלות הראשון משקלו לטרא השני משקלו ג' לטרין השלישי משקלו ט' לטרי' החמשי פ"א ליטרין הרביעי כ"ז לטרין | ||
| + | |- | ||
| + | | | ||
| + | ::Do as follows: first write 1, which is the weight of the first, like this: | ||
| + | ::<math>\scriptstyle{\color{blue}{a_1=1}}</math> | ||
| + | |style="text-align:right;"|וככה תעשה כתוב בתחילה א' משקלו של משקל הראשון ואח"כ כתוב כך | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color: blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |1 | ||
| + | |- | ||
| + | |1 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |א | ||
| + | |- | ||
| + | |א | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Sum them together; it is 2. Add another 1 to it; it is 3 and this is the second weight. | ||
| + | ::<math>\scriptstyle{\color{blue}{a_2=\left(1+1\right)+1=2+1=3}}</math> | ||
| + | |style="text-align:right;"|חברם יחד ויהיה ב' תוסיף עוד א' עליה ויהיה ג' זהו משקל השני | ||
| + | |- | ||
| + | | | ||
| + | ::Then, write like this: | ||
| + | |style="text-align:right;"|אח"כ כתוב כך | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color: blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |1 | ||
| + | |- | ||
| + | |1 | ||
| + | |- | ||
| + | |3 | ||
| + | |- | ||
| + | |3 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |א | ||
| + | |- | ||
| + | |א | ||
| + | |- | ||
| + | |ג | ||
| + | |- | ||
| + | |ג | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Sum them together; it is 8. Add another one; it is 9 and this is the third weight. | ||
| + | ::<math>\scriptstyle{\color{blue}{a_3=\left(1+1\right)+\left(3+3\right)+1=8+1=9}}</math> | ||
| + | |style="text-align:right;"|חברם יחד ויהיה ח' תוסיף עוד אחת ויהיה ט' זהו משקל השלישי | ||
| + | |- | ||
| + | | | ||
| + | ::Then, write like this: | ||
| + | |style="text-align:right;"|אח"כ כתוב כך | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color: blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |1 | ||
| + | |- | ||
| + | |1 | ||
| + | |- | ||
| + | |3 | ||
| + | |- | ||
| + | |3 | ||
| + | |- | ||
| + | |9 | ||
| + | |- | ||
| + | |9 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |א | ||
| + | |- | ||
| + | |א | ||
| + | |- | ||
| + | |ג | ||
| + | |- | ||
| + | |ג | ||
| + | |- | ||
| + | |ט | ||
| + | |- | ||
| + | |ט | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Sum them together; it is 26. Add another one; it is 27 and this is the fourth weight. | ||
| + | ::<math>\scriptstyle{\color{blue}{a_4=\left(1+1\right)+\left(3+3\right)+\left(9+9\right)+1=26+1=27}}</math> | ||
| + | |style="text-align:right;"|חברם יחד ויהיה כ"ו תוסיף עוד אחת ויהיה כ"ז זהו משקל רביעי | ||
| + | |- | ||
| + | | | ||
| + | ::Then, write like this: | ||
| + | |style="text-align:right;"|אח"כ כתוב כך | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color: blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |1 | ||
| + | |- | ||
| + | |1 | ||
| + | |- | ||
| + | |3 | ||
| + | |- | ||
| + | |3 | ||
| + | |- | ||
| + | |9 | ||
| + | |- | ||
| + | |9 | ||
| + | |- | ||
| + | |27 | ||
| + | |- | ||
| + | |27 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |א | ||
| + | |- | ||
| + | |א | ||
| + | |- | ||
| + | |ג | ||
| + | |- | ||
| + | |ג | ||
| + | |- | ||
| + | |ט | ||
| + | |- | ||
| + | |ט | ||
| + | |- | ||
| + | |זב | ||
| + | |- | ||
| + | |זב | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Sum them together; it is 88. Add another one; it is 81 and this is the fifth weight. | ||
| + | |style="text-align:right;"|חברם יחד ויהיה פ' תוסיף עוד אחת ויהיה פ"א זהו משקל חמישי | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | ::<math>\scriptstyle{\color{blue}{a_5=\left(1+1\right)+\left(3+3\right)+\left(9+9\right)+\left(27+27\right)+1=80+1=81}}</math> | ||
| + | |- | ||
| + | | | ||
| + | ::The total is 121. | ||
| + | ::<math>\scriptstyle{\color{blue}{a_1+a_2+a_3+a_4+a_5=1+3+9+27+81=121}}</math> | ||
| + | |style="text-align:right;"|ס"ה קכ"א | ||
| + | |- | ||
| + | | | ||
| + | ::You can keep adding this way. | ||
| + | |style="text-align:right;"|ועל דרך זה תוכל להוסיף | ||
| + | |- | ||
| + | | | ||
| + | ::Or, we use this way: for the first weight take one; for the second 3 times 1; for the third 3 times 3; for the fourth 3 times 9; for the fifth 3 times 27 and so on - your sign is 3, meaning 3 times the first weight. | ||
| + | |style="text-align:right;"|או נלך אצל דרך זה למשקל הראשו' קח את אחת לשנים גפ"א לשלישי' ג'פג' לרביעי' גפ"ט לחמישי' ג"פ כ"ז וכן לעולם וסימניך ושלישי' על כולו פי' ג"פ משקל הראשון | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{\begin{cases}\scriptstyle a_1 =1\\\scriptstyle a_2=3\sdot a_1=3\sdot1=3\\\scriptstyle a_3=3\sdot a_2=3\sdot3=9\\\scriptstyle a_4=3\sdot a_3=3\sdot9=27\\\scriptstyle a_5=3\sdot a_4=3\sdot27=81\\\scriptstyle a_i=3\sdot a_{i-1}\end{cases}}}</math> | ||
| + | | | ||
| + | |- | ||
| + | ! | ||
| + | |||
| + | ==== <span style=color:Green>Divide a Quantity - Money</span> ==== | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:money|644|8Dxu}}18) If you want to divide 5 pešiṭim to one third and one quarter so that nothing remains | ||
| + | :<math>\scriptstyle\frac{1}{3}X+\frac{1}{4}X=5</math> | ||
| + | |style="text-align:right;"|<big>י"ח אם</big> תרצה לחלק ה"פ לשליש ולרביע ולא ישאר מהן כלום{{#annotend:8Dxu}} | ||
| + | |- | ||
| + | | | ||
| + | ::First, say: where are a third and a quarter found? In 12: the third is 4 and the quarter is 3. Sum them together; it is 7 and this is the denominator. | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(\frac{1}{3}\sdot12\right)+\left(\frac{1}{4}\sdot12\right)=4+3=7}}</math> | ||
| + | |style="text-align:right;"|אמור תחילה שליש ורביע בכמה ימצאון בי"ב השליש הוא ד' הרביע הוא ג' חברם יחד הרי ז' וזהו המורה | ||
| + | |- | ||
| + | | | ||
| + | ::<span style=color:Green>'''Rule of Three:'''</span> if you want to know how much is due to the one who has the third, which is 4, multiply 4 by 5 pešiṭim; the result is 20. Divide it by 7; you find 2 times 7 in it and an excess of 6 parts, of which 7 is a whole unit. So, he has 2 whole pešiṭim and 6 parts of which 7 is a whole unit. | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{1}{3}X=\frac{4\sdot5}{7}=\frac{20}{7}=2+\frac{6}{7}}}</math> | ||
| + | |style="text-align:right;"|ואם תרצה לידע בכמה מגיע לזה שיש לו השליש שהוא ד' כפול ד' בה' פשי' ויעלה כ' תחלק בז' ותמצא בו ב' פ"ז ועודף ו' חלקי' שז' מהן חלק שלם הרי שיש לזה ב' פשי' שלימי' וו' שברי' שז' מהן חלק שלם | ||
| + | |- | ||
| + | | | ||
| + | ::For the one who has a quarter: multiply 3 by 5; the result is 15. Divide it by 6; the result is 2 pešiṭim and one part of which 7 is a whole pešuṭ. | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{1}{4}X=\frac{3\sdot5}{7}=\frac{15}{7}=2+\frac{1}{7}}}</math> | ||
| + | |style="text-align:right;"|ומי שיש לו הרביעי כפול ג' בה' ויעלה ט"ו תחלק ממנו ו' ויעלה ב' פשי' וחלק אחד שז' פשי' שלם | ||
| + | |- | ||
| + | | | ||
| + | ::Thus, the 5 pešuṭim are divided into a third and a quarter with no remainder. | ||
| + | |style="text-align:right;"|הרי שנחלקו ה' [פשוטים] ‫<ref>123v</ref>לג' ולד' ולא נשאר מהן כלום | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:money|644|k9es}}Likewise, if you have 12 and you wish to divide them to a third, a half, and a quarter, so that nothing remains | ||
| + | :<math>\scriptstyle\frac{1}{2}X+\frac{1}{3}X+\frac{1}{4}X=12</math> | ||
| + | |style="text-align:right;"|וכן אם בידך י"ב פשי' ותרצה לחלקם לחצאין לשליש ולרביעי ולא ישאר כלום{{#annotend:k9es}} | ||
| + | |- | ||
| + | | | ||
| + | ::Say: where are a half, a third and a quarter found? In 12: the half is 6, the third is 4 and the quarter is 3. Sum them together; it is 13 and this is the denominator. | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(\frac{1}{2}\sdot12\right)+\left(\frac{1}{3}\sdot12\right)+\left(\frac{1}{4}\sdot12\right)=6+4+3=13}}</math> | ||
| + | |style="text-align:right;"|אמור חצי שליש ורביע אָנה ימצאון בי"ב החצי ו' השליש ד' הרביע ג' צרוף יחד הרי י"ג וזהו המורה | ||
| + | |- | ||
| + | | | ||
| + | ::<span style=color:Green>'''Rule of Three:'''</span> multiply 6, which is the half, by 12; the result is 72. Divide it, meaning how many times 13 is in it; you find 5 times and 7 parts of which 13 is a whole unit. | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{1}{2}X=\frac{6\sdot12}{13}=\frac{72}{13}=5+\frac{7}{13}}}</math> | ||
| + | |style="text-align:right;"|אח"כ כפול ו' שהוא החציה בי"ב <s>וע</s> ויעלה ע"ב תחלק כמה פעמי' יש בו י"ג ותמצא ה' פעמי' וז' שברי' שי"ג מהן פשי' שלם | ||
| + | |- | ||
| + | | | ||
| + | ::Multiply the third, which is 4, by 12; the result is 48. Divide it by 13; you find 3 and 9 parts of 13. | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{1}{3}X=\frac{4\sdot12}{13}=\frac{48}{13}=3+\frac{9}{13}}}</math> | ||
| + | |style="text-align:right;"|אח"כ כפול השליש שהוא ד' בי"ב ויעלה מ"ח תחלק ממנו י"ג תמצא ג' וט' שברי' מי"ג | ||
| + | |- | ||
| + | | | ||
| + | ::Multiply 3 by 12; the result is 36. Divide it by 13; you find 2 and 10 parts of 13. | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{1}{4}X=\frac{3\sdot12}{13}=\frac{36}{13}=2+\frac{10}{13}}}</math> | ||
| + | |style="text-align:right;"|וכן כפול ג' בי"ב ויעלה ל"ו חלק מהן י"ג ותמצא ב' וי' שברי' מי"ג | ||
| + | |- | ||
| + | | | ||
| + | ::And so on. | ||
| + | |style="text-align:right;"|וכן לעולם | ||
| + | |- | ||
| + | ! | ||
| + | |||
| + | ==== <span style=color:Green>Find a Quantity Problem - Whole from Parts - Fish</span> ==== | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:fish|650|iHCN}}19) You have a fish whose body weighs 10 liṭra and its tail and head weigh a third, a quarter, a fifth, and a sixth of its whole. How much is the weight of the whole [fish]? | ||
| + | :<math>\scriptstyle\frac{1}{3}X+\frac{1}{4}X+\frac{1}{5}X+\frac{1}{6}X+10=X</math> | ||
| + | |style="text-align:right;"|<big>י"ט הרי</big> יש לפניך דג שמשקל גופו <s>י'</s> י' לטראות ומשקל זנבו ראשו שלישיתו רבי' חמי' ששי' כמה כל משקלו{{#annotend:iHCN}} | ||
| + | |- | ||
| + | | | ||
| + | ::Answer: find a number divisible by 3, 4, 5, and 6; it is 60. | ||
| + | |style="text-align:right;"|תשובה מנה מספר המתחלק לגדה"ו וזהו ס‫' | ||
| + | |- | ||
| + | | | ||
| + | ::When you subtract 3, 4, 5, and 6 from 60, i.e. its third, quarter, fifth, and sixth; you sum them together; the result is 57 and the remainder is 3; and this is the denominator. | ||
| + | |style="text-align:right;"|וכשתסיר מס' גדה"ו פי' השליש והרבי' והחומש והשישי' ותצרפם יחד ויעלה נ"ז ועודף ג' זהו המורה | ||
| + | |- | ||
| + | |colspan="2"| | ||
| + | ::<math>\scriptstyle{\color{blue}{60-\left[\left(\frac{1}{3}\sdot60\right)+\left(\frac{1}{4}\sdot60\right)+\left(\frac{1}{5}\sdot60\right)+\left(\frac{1}{6}\sdot60\right)\right]=60-57=3}}</math> | ||
| + | |- | ||
| + | | | ||
| + | ::Multiply 10 by 60; the result is 600. | ||
| + | |style="text-align:right;"|כפול י' בס' ויעלה ת"ר | ||
| + | |- | ||
| + | | | ||
| + | ::Divide it by 3; you find in it two hundred times 3. | ||
| + | |style="text-align:right;"|תחלק ממנו ג' ותמצא בו ר' פעמי' ג‫' | ||
| + | |- | ||
| + | | | ||
| + | ::So, the weight of the whole fish you asked about is two hundred liṭra. | ||
| + | ::<math>\scriptstyle{\color{blue}{X=\frac{10\sdot60}{3}=\frac{600}{3}=200}}</math> | ||
| + | |style="text-align:right;"|וכן כל משקלו של דג ששאלנו מאתם | ||
| + | |- | ||
| + | ! | ||
| + | |||
| + | ==== <span style=color:Green>Multiple Quantities - Selling Cloth</span> ==== | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:selling cloth|652|evCI}}20) Question: a man gave his three sons [30] cubits of cloth to sell. One sold one cubit for 4 zehuvim, the second for 5 zehuvim, and the third for 6 zehuvim. All of them earned the same amount of money. | ||
| + | :How much money did each of them get from the sale and how many cubits did each of them sell? | ||
| + | |style="text-align:right;"|<big>כ' שאלה</big> אדם נתן לג' בניו [ל'] אמות בגד למכור והאחד מכר האמה עבור ד' זהו' והשני עבור ה' והג' עבור ו' זהו' וכולם מביאי' מעות שוות זה כזה<br> | ||
| + | חוד כמה מעות להג' מן המקח וכמה אמות מכר כל אחד{{#annotend:evCI}} | ||
| + | |- | ||
| + | | | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle X+Y+Z=30\\\scriptstyle4X=5Y=6Z\end{cases}</math> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | ::Answer: first I will show you how much does the one who gives one cubit for 4 zehuvim have: | ||
| + | |style="text-align:right;"|תשובה בתחילה אראך כמה שהיה לזה שנתן אמה עבור ד' זהו‫' | ||
| + | |- | ||
| + | | | ||
| + | ::First, say: in [which number] there are a quarter, a fifth, and a sixth? | ||
| + | |style="text-align:right;"|אמור תחילה <s>ב</s> רביעי' חמישי' ששית אנה ימצאון | ||
| + | |- | ||
| + | | | ||
| + | ::In 60: the quarter is 15; the fifth is 12; the sixth is 10. Sum them together; it is 37 and this is the denominator. | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(\frac{1}{4}\sdot60\right)+\left(\frac{1}{5}\sdot60\right)+\left(\frac{1}{6}\sdot60\right)=15+12+10=37}}</math> | ||
| + | |style="text-align:right;"|בס' רביעי' ט"ו חמישי' י"ב ששות י' חברם יחד ויהיה ל"ז זהו המורה | ||
| + | |- | ||
| + | | | ||
| + | ::Then, mulitply 15, which is the quarter, by 30, which is the measure of the cloth; you find it is 450. | ||
| + | |style="text-align:right;"|ואח"כ כפול הט"ו שהוא הרביעי' בל' כמדת הבגד ותמצא ת"נ | ||
| + | |- | ||
| + | | | ||
| + | ::Divide it by 37; you find in it 12 times 37 and six parts of 37. | ||
| + | ::<math>\scriptstyle{\color{blue}{X=\frac{15\sdot30}{37}=\frac{450}{37}=12+\frac{6}{37}}}</math> | ||
| + | |style="text-align:right;"|תחלק ת"ן לל"ז ותמצא בו י"ב פעמי' ל"ז וששה חלקי' של"ז אמה שלימה נמצא שהיה לזה שנתן אמה עבור ד' זהו' י"ב אמות וששה חלקי' של"ז מהן אמה שלימה | ||
| + | |- | ||
| + | | | ||
| + | ::If you wish to know how much money does the one who gives one cubit for 4 zehuvim have: | ||
| + | |style="text-align:right;"|ואם תחפוץ לידע כמה מעות גבהו לזה שנתן אמה עבור ד' זהובי‫' | ||
| + | |- | ||
| + | | | ||
| + | ::He has 12 cubits and six parts of 37 of a cubit. | ||
| + | |style="text-align:right;"|‫<ref>124r</ref>י"ב אמות היו לו וששה חלקים של"ז מהן אמה שלימה | ||
| + | |- | ||
| + | | | ||
| + | ::12 times 4 is 48; and for the 6 parts do as follows: | ||
| + | |style="text-align:right;"|הרי י"ב ד"פ י"ב עולה מ"ח ומן הו' חלקי' הנותרי' עשה כך | ||
| + | |- | ||
| + | | | ||
| + | ::Divide each gold coin into 7 tapuḥim and say: if these were 6 parts of 37, i.e. the price of one cubit of cloth, then their price were 28 tapuḥim. Now that they are only 6, for how much are they? | ||
| + | |style="text-align:right;"|חלוק כל זהב בז' תפוחי' ותאמר כך אילו היו הו' חלקי' ל"ז [דהיינו דמיהם של בגד אמה] אז היה דמיהן כ"ח תפוחי' עתה שהם רק ו' כמה הם | ||
| + | |- | ||
| + | | | ||
| + | ::Multply 6 by 28; the result is 168. | ||
| + | |style="text-align:right;"|אז כפול בכ"ח [ויעלה ח'ו'א'‫] | ||
| + | |- | ||
| + | | | ||
| + | ::Divide it by 37; you find in it 4 times 37 and twenty parts of 37 of a whole tapuaḥ. | ||
| + | |style="text-align:right;"|וחלוק אותו לל"ז ותמצא בו ד' פעמי' ל"ז [ועשרים חלקים] של"ז עושי' תפוח שלם | ||
| + | |- | ||
| + | | | ||
| + | ::We find that he charge 48 zehuvim, 4 tapuḥim and twenty parts of 37 of a whole tapuaḥ. | ||
| + | |style="text-align:right;"|נמצא שזה גבה מ"ח <s>תפוחי'</s> זהובי' וד' תפוחי' ועשרי' חלקי' של"ז תפוח שלם | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | ::<math>{\color{blue}{\begin{align}\scriptstyle4X &\scriptstyle=4\sdot\left(12+\frac{6}{37}\right)=\left(4\sdot12\right)+\left(4\sdot\frac{6}{37}\right)=48+\frac{\frac{28\sdot6}{37}}{7}=48+\frac{\frac{168}{37}}{7}=48+\frac{4+\frac{20}{37}}{7}\\&\scriptstyle=48+\frac{4}{7}+\frac{\frac{20}{37}}{7}\\\end{align}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | ::To know how many cubits does the one who gives one cubit for 5 zehuvim sell do as follows: | ||
| + | |style="text-align:right;"|לדעת כמה אמו' מכר זה שנתן אמה עבור ה' זהו' עשה כך | ||
| + | |- | ||
| + | | | ||
| + | ::Multiply the fifth of a sixth, meaning 12, by the number of the cubits of the cloth, which is 30; the result is 360. | ||
| + | |style="text-align:right;"|כפול החומש משישית דהיינו י"ב במניין אמו' הבגד שהם ל' [ויעלה 0'ו'ג'‫] | ||
| + | |- | ||
| + | | | ||
| + | ::Divide it by 37, which is the denominator; you find in it 9 cubits and 27 parts of 37 of a whole cubit. | ||
| + | ::<math>\scriptstyle{\color{blue}{Y=\frac{12\sdot30}{37}=\frac{360}{37}=9+\frac{27}{37}}}</math> | ||
| + | |style="text-align:right;"|ותחלק ממנו ל"ז [שהוא] המורה ותמצא בו ט' אמות וכ"ז חלקי' של"ז מהן עושי' אמה שלמה | ||
| + | |- | ||
| + | | | ||
| + | ::If you want to know how much money he charges for the 9 cubits, meaning 45 zehuvim, and 27 parts: | ||
| + | |style="text-align:right;"|ואם תחפוץ לידע כמה מעות גבה ט' אמות היינו מ"ה זהו' וכ"ז חלקי‫' | ||
| + | |- | ||
| + | | | ||
| + | ::If they were 37, they would have been sold for 35 tapuḥim, considering each zehuv as 7 tapuḥim as above. Now that they are only 27, multiply 27 by 35; the result is 945. Divide it by 37; you find 25 tapuḥim and 20 parts of which 37 are a whole tapuḥ. | ||
| + | |style="text-align:right;"|אילו היה ל"ז היו נמכרי' בעד ל"ה תפ<s>י</s>וחי' כשתעריך כל זהו' בעד ז' תפוחי' כדלעיל עכשיו שהוא רק כ"ז [כמה כפול כ"ז] בל"ה [ויעלה הד"ט] ותחלק ממנו ל"ז ותמצא כ"ה תפוחי' וך' חלקי' של"ז עושין תפוח שלם | ||
| + | |- | ||
| + | | | ||
| + | ::Convert 21 tapuḥim into 3 zehuvim and add them to 45; it is 48 and you are left with 4 tapuḥim and 20 parts of which 37 are a whole tapuḥ. | ||
| + | |style="text-align:right;"|עשה מכ"א [.] תפוחי' ג' זהו' וחברם יחד עם מ"ה ויהיה מ"ח ויוותרו לך ד' תפוחי' וך' חלקי' של"ז תפוח שלם | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | ::<math>{\color{blue}{\begin{align}\scriptstyle5Y &\scriptstyle=5\sdot\left(9+\frac{27}{37}\right)=\left(5\sdot9\right)+\left(5\sdot\frac{27}{37}\right)=45+\frac{\frac{35\sdot27}{37}}{7}=45+\frac{\frac{945}{37}}{7}=45+\frac{25+\frac{20}{37}}{7}\\&\scriptstyle=45+\frac{21+4+\frac{20}{37}}{7}=45+3+\frac{4}{7}+\frac{\frac{20}{37}}{7}=48+\frac{4}{7}+\frac{\frac{20}{37}}{7}\\\end{align}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | ::We find that the money of the first is the same as the [money] of the second. | ||
| + | |style="text-align:right;"|נמצא שמעו' הראשונות כשיני | ||
| + | |- | ||
| + | | | ||
| + | ::To know the amount of money and cubits of the one who sells a cubit for 30 zehuvim: multiply a sixth of 60 by 30, which is the number of the cubits; the result is 300. Divide the result by 37; you find 8 cubits and 4 parts of which 37 are one unit. | ||
| + | ::<math>\scriptstyle{\color{blue}{Z=\frac{10\sdot30}{37}=\frac{300}{37}=8+\frac{4}{37}}}</math> | ||
| + | |style="text-align:right;"|לדעת המעו' ואמו[ת]‫<ref>M ואמור</ref> המוכר אמה עבור ל' זהו' כפול חלק ששי' מס' בל' מניין האמות [דהיינו ל' ויעלה 0'0'ג'] ומן העולה תחלוק ל"ז ותמצא ח' אמות וד' חלקי' של"ז עושי' חלק שלם | ||
| + | |- | ||
| + | | | ||
| + | ::To know how much is his money: 8 times 6 zehuvim are 48. As for the 4 remaining parts: if they were 37, they would have been sold for 42 tapuḥim. Now that they are only 4, multiply 4 by 42; the result is 168. Divide the result by 37 zehuvim; you find 4 tapuḥim and 20 parts of which 37 is a whole tapuḥ. | ||
| + | |style="text-align:right;"|ולדעת כמה מעותיו ח' פעמי' ו' זהו' היינו מ"ח ד' חלקי' הנותרי' אילו היה ל"ז היו נמכרי' במ"ב תפוחי' עתה שהם רק ד' בכמה כפול ד' במ"ב [ויעלה חו"א] ומן העולה תחלק [ל"ז] זהו' ותמצא ד' תפוחי' וך' חלקי' של"ז תפוח שלם | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | ::<math>{\color{blue}{\begin{align}\scriptstyle6Z &\scriptstyle=6\sdot\left(8+\frac{4}{37}\right)=\left(6\sdot8\right)+\left(6\sdot\frac{4}{37}\right)=48+\frac{\frac{42\sdot4}{37}}{7}=48+\frac{\frac{168}{37}}{7}\\&\scriptstyle=48+\frac{4+\frac{20}{37}}{7}=48+\frac{4}{7}+\frac{\frac{20}{37}}{7}\\\end{align}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | ::We find that there is no [difference] between them regarding the amount of money. | ||
| + | |style="text-align:right;"|נמצא שאין בין זה לזה [במנין]‫<ref>M כמבין</ref> המעות | ||
| + | |- | ||
| + | | | ||
| + | ::The first sold 12 cubit plus 6 parts; the second sold 9 cubits and 27 parts; the third sold 8 cubits and 4 parts. | ||
| + | |style="text-align:right;"|והראשון מכר י"ב אמו' יותר ו' חלקי' ושני מכר ט' אמו' וכ"ז חלקי' והשלישי מכר ח' אמו' וד' חלקי‫' | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|וזהו סימנם | ||
| + | |- | ||
| + | ! | ||
| + | |||
| + | ==== <span style=color:Green>Partnership Problems - for the Same Time - Three Partners</span> ==== | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:three partners|661|fU09}}21) Question: three friends want to donate together as a group 40 liṭra zehuvim. The first has gold that worth 3 zehuvim per liṭra, the second [has gold that] worth 5 zehuvim [per liṭra], and the third has gold that worth 8 zehuvim per liṭra. How many liter does each of them has equally? | ||
| + | :<math>\scriptstyle\frac{1}{3}X+\frac{1}{5}X+\frac{1}{8}X=40</math> | ||
| + | |style="text-align:right;"|<big>כ"א ועוד</big> שאלה ג' חבירי' רצו לתת בחבורה מ' לטרי' זהו' לראשון יש לו זהב ששוה הלטרי' ג' זהו' לשני ששוה ה' זהו' ולשלישי יש לו זהב ששוה הלטר' ח' זהו‫'<br> | ||
| + | כמה יש כל אחד לטרי' שוה בשוה{{#annotend:fU09}} | ||
| + | |- | ||
| + | | | ||
| + | ::Answer: find a number divisible by 3, 5, and 8: multiply 3 by 5; it is 15. Multiply 8 by 15; the result is 120. | ||
| + | ::<math>\scriptstyle{\color{blue}{3\sdot5\sdot8=15\sdot8=120}}</math> | ||
| + | |style="text-align:right;"|תשובה עשה מספ' המתחלק לגה"ח כיצד כפול ג' על ה' הרי ט"ו כפול ח' על ט"ו ויעלה ק"כ | ||
| + | |- | ||
| + | | | ||
| + | ::Take from 120 its third, it fifth, and its eighth, and add them up; it is 79 and this is the denominator. | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(\frac{1}{3}\sdot120\right)+\left(\frac{1}{5}\sdot120\right)+\left(\frac{1}{8}\sdot120\right)=79}}</math> | ||
| + | |style="text-align:right;"|הסר מק"כ <s>ה</s> השליש והחומש [שמינית] וחבר יחד ויהיה ע"ט וזה המור‫' | ||
| + | |- | ||
| + | | | ||
| + | ::To know how many liṭra the one [whose gold] is worth 3 zehuvim should contribute: take a third of 120; it is 40. Multiply 40 by 40, which is the number of the liṭra and divide the result by 79. | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{1}{3}X=\frac{\left(\frac{1}{3}\sdot120\right)\sdot40}{79}=\frac{40\sdot40}{79}}}</math> | ||
| + | |style="text-align:right;"|לדעת כמה לטרי' צריך לשום זה שזה שוה ג' זהו' קח השליש מק"כ ‫<ref>124v</ref>וזהו מ' כפול מ' על מ' מניין הליטרין ומן העולה תחלק ע"ט | ||
| + | |- | ||
| + | | | ||
| + | ::The same for all of them: | ||
| + | |style="text-align:right;"|וכן לכולם | ||
| + | |- | ||
| + | | | ||
| + | ::For the second: multiply the fifth of 120 by 40. | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{1}{5}X=\frac{\left(\frac{1}{5}\sdot120\right)\sdot40}{79}}}</math> | ||
| + | |style="text-align:right;"|לשני כפול החומש מק"ך במ‫' | ||
| + | |- | ||
| + | | | ||
| + | ::For the third: multiply the [eighth] of 120 by 40. | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{1}{8}X=\frac{\left(\frac{1}{8}\sdot120\right)\sdot40}{79}}}</math> | ||
| + | |style="text-align:right;"|לשלישי' כפול מק"כ | ||
| + | |- | ||
| + | | | ||
| + | :I have already elaborated on this matter. | ||
| + | |style="text-align:right;"|כמה כבר הארכתי בעניין זה | ||
| + | |- | ||
| + | ! | ||
| + | |||
| + | ==== <span style=color:Green>Multiple Quantities</span> ==== | ||
| + | | | ||
| + | |- | ||
| + | !<span style=color:Green>Four Coins</span> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:four coins|652|LwaJ}}22) Question: you have four coins that are worth 40 pešuṭim: the first is equal to a half and a sixth of the second. What is left from the second equals two-thirds of the third. The third is equal to a third, a quarter, and a sixth of the first. The fourth is equal to four-fifths of the [third]. How much does each [of the coins] worth? | ||
| + | |style="text-align:right;"|<big>כב הרי</big> שיש לפניך ד' מטבעות ששוים מ' פשי‫'<br> | ||
| + | הראשונה שוה החצי והשתות מן השנייה<br> | ||
| + | והנשאר מן השני הוא ב' שלישי מן השלישי‫'<br> | ||
| + | והשלישי' שוה שליש ורביע ושתות מן הראשונ‫'<br> | ||
| + | והרביעי' שוה ד' חומשי' מן הב‫'<br> | ||
| + | לדעת כמה שוה כל אחת מחברת' בקיצור{{#annotend:LwaJ}} | ||
| + | |- | ||
| + | | | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle\sum_{i=1}^4 a_i =40\\\scriptstyle a_1=\left(\frac{1}{2}+\frac{1}{6}\right)\sdot a_2\\\scriptstyle a_2-\left[\left(\frac{1}{2}+\frac{1}{6}\right)\sdot a_2\right]=a_2-a_1=\frac{2}{3}a_3\\\scriptstyle a_3=\left(\frac{1}{3}+\frac{1}{4}+\frac{1}{6}\right)\sdot a_1\\\scriptstyle a_4=\frac{4}{5}\sdot{\color{red}{a_3}}\end{cases}</math> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | ::Do as follows: | ||
| + | |style="text-align:right;"|עשה כך | ||
| + | |- | ||
| + | | | ||
| + | ::For the first that is equal to a half and a sixth of the second: in which number there are a whole half and a whole sixth? In six: the half is 3 and the sixth is 1. So it is 4 of 6, as if it is said: the first is 2-thirds of the second. | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(\frac{1}{2}\sdot6\right)+\left(\frac{1}{6}\sdot6\right)=3+1=4\longrightarrow a_1=\frac{2}{3}a_2}}</math> | ||
| + | |style="text-align:right;"|הראשונה ששוה החצי והשתו' מן השנייה באיזה מספר נמצאי' חצי ושתות שלמי' בששה החצי מהחצי ג' והשתות א' הרי ד' מן הוי' כאילו אמר הראשונ' ב' שליש מן השני | ||
| + | |- | ||
| + | | | ||
| + | ::For the third that is equal to a third, a quarter, and a sixth of the first: in which number these fractions are found? In 12: the third is 4; the quarter is 3; the sixth is 2. Sum them together; it is 9, as if it is said: the third is 3-quarters of the first. | ||
| + | |style="text-align:right;"|ושלישית‫<ref>M ושליש</ref> ששוה [שליש]‫<ref>M רביע</ref> ורביע ושתות מן הראשון אילו החלקי' בכמה נמצאון בי"ב השליש ד' הרביע ג' השתות ב' כלול יחד הרי ט' הוי כאילו אמר השליש ג' רבי' מן הראשונה | ||
| + | |- | ||
| + | |colspan="2"| | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(\frac{1}{3}\sdot12\right)+\left(\frac{1}{4}\sdot12\right)+\left(\frac{1}{6}\sdot12\right)=4+3+2=9\longrightarrow a_3=\frac{9}{12}a_1=\frac{3}{4}a_1}}</math> | ||
| + | |- | ||
| + | | | ||
| + | ::For the fourth that is equal to 4-fifths of the [third]: there is no need for an explanation, because it is well explained. | ||
| + | ::<math>\scriptstyle{\color{blue}{a_4=\frac{4}{5}{\color{red}{a_3}}}}</math> | ||
| + | |style="text-align:right;"|‫[והד']‫<ref>M והה'</ref> ששוה ד' חומשי' מן (מן) השני אין צריך ביאור דהא מבואר היטב | ||
| + | |- | ||
| + | | | ||
| + | ::To know how you find the fractions, understand the fractions we mentioned in the question: a half and a sixth - wherever you find a sixth, you find also a third; we also mentioned a quarter and a fifth. All these fractions are found in sixty. Hence, the second coin is 60. | ||
| + | ::<math>\scriptstyle{\color{blue}{b_2=60}}</math> | ||
| + | |style="text-align:right;"|לדעת איך תמצאם החלקי' חלוקי' בפי' הבן איזה חלקי' הזכרנו בשאל' חצי ושתות ובכל מקום שתמצא שתות שליש גם תמצא גם הוזכרנו רביעי' וחומש וכל אילו החלקי' נמצא בששי' נמצאת מטבע שנייה ס‫' | ||
| + | |- | ||
| + | | | ||
| + | ::The half and the sixth are 30 and 10. Add them together; it is 40 and this is the first coin. | ||
| + | ::<math>\scriptstyle{\color{blue}{b_1=\left(\frac{1}{2}+\frac{1}{6}\right)\sdot b_2=\left(\frac{1}{2}\sdot60\right)+\left(\frac{1}{6}\sdot60\right)=30+10=40}}</math> | ||
| + | |style="text-align:right;"|החצי והשתות גם ל' וי' {{#annot:term|178,1552|DAsZ}}כללם יחד{{#annotend:DAsZ}} הרי מ' זו היא מטבע הראשונה | ||
| + | |- | ||
| + | | | ||
| + | ::By how much 60 exceeds over 40? by 20 and this is what we said in the question "what is left from the second", which exceeds the first, "is two-thirds of the third". So, the third coin is 30, because when you take a third, a quarter, and a sixth of 40, you find the third. | ||
| + | |style="text-align:right;"|כמה עודף ס' יותר על מ' כ' זהו' שאמרנו בשאלה והנשאר מן השני עודף על הראשון הוא שני שליש מן השלישי' כי מטבע [שלישי ל']‫<ref>M שלישיתו</ref> כשתסיר גד"ו פי' שליש רביעי' שישית ממ' תמצא שלישי | ||
| + | |- | ||
| + | |colspan="2"| | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{2}{3}b_3=b_2-b_1=60-40=20\longrightarrow b_3=\left(\frac{1}{3}+\frac{1}{4}+\frac{1}{6}\right)\sdot b_1=\left(\frac{1}{3}+\frac{1}{4}+\frac{1}{6}\right)\sdot40=30}}</math> | ||
| + | |- | ||
| + | | | ||
| + | ::To know the fourth coin, divide [30] into 5 parts. We find 5 times 6. So, 4-fifths of 30 is 24. | ||
| + | ::<math>\scriptstyle{\color{blue}{b_4=\frac{4}{5}\sdot b_3=\frac{4}{5}\sdot30=\frac{4}{5}\sdot5\sdot6=24}}</math> | ||
| + | |style="text-align:right;"|לדעת מטבע רביעי' תחלק לה' חלקי' נמצא ה' פעמי' ו' ד' חומשי' מל' הרי כ"ד | ||
| + | |- | ||
| + | | | ||
| + | ::This is their order: 40, 60, 30, 24. Sum them together; it is 154 and this is the denominator. | ||
| + | ::<math>\scriptstyle{\color{blue}{b_1+b_2+b_3+b_4=40+60+30+24=154}}</math> | ||
| + | |style="text-align:right;"|וזהו סדורן מ' ס' ל' כ"ד צרוף אותם יחד הרי קנ"ד זהו המורה את החידה | ||
| + | |- | ||
| + | | | ||
| + | ::To know how much is the value of the first coin, multiply 40 by 40, which is the value of the coins and divide the result by 154. You find its diagram like this: | ||
| + | |style="text-align:right;"|לדעת כמה מטבע ראשונה למלאו' מניין ארבעי' כפול מ' במ' שהוא מניין המעות ומן העולה תחלק קנ"ד ותמצא צורתו כך | ||
| + | |- | ||
| + | | | ||
| + | ::Meaning 10 pešiṭim and sixty parts of which 154 are a whole pašuṭ and this is the value of the first coin. | ||
| + | ::<math>\scriptstyle{\color{blue}{a_1=\frac{40\sdot40}{154}=10+\frac{60}{154}}}</math> | ||
| + | |style="text-align:right;"|פי' י' פשי' וששי' [חלקים] שקנ"ד פשי' שלם זהו סך מטבע ראשונ‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Multiply 60 by 40 and divide the result by 154. Its diagram is like this: | ||
| + | |style="text-align:right;"|אח"כ כפול ס' במ' ומן העולה תחלק קנ"ד ויהיה צורתו כך | ||
| + | |- | ||
| + | | | ||
| + | ::Meaning 15 pešiṭim and 90 parts of which 154 are a whole pašuṭ and this is the value of the second coin. | ||
| + | ::<math>\scriptstyle{\color{blue}{a_2=\frac{60\sdot40}{154}=15+\frac{90}{154}}}</math> | ||
| + | |style="text-align:right;"|פי' ט"ו פשי' וצ' חלקי' שקנ"ד פשי' שלם זהו סך מטבע שנייה | ||
| + | |- | ||
| + | | | ||
| + | ::Multiply 30 by 40 and divide the result by 154. Its diagram is like this: | ||
| + | |style="text-align:right;"|אח"כ כפול ל' על מ' וחלקהו בקנ"ד ויהיה צורתו כך | ||
| + | |- | ||
| + | | | ||
| + | ::Meaning 7 pešiṭim and 122 parts of which 154 are a whole pašuṭ and this is the value of the third coin. | ||
| + | ::<math>\scriptstyle{\color{blue}{a_3=\frac{30\sdot40}{154}=7+\frac{122}{154}}}</math> | ||
| + | |style="text-align:right;"|פי' ז' פשי' קכ"ב חלקי' שקנ"ד פשי' שלם זהו סך מטבע ג‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Multiply 24 by 40 and divide the result by 154. Its diagram is like this: | ||
| + | |style="text-align:right;"|אח"כ כפול כ"ד במ' ומן העולה תחלק קנ"ד וצורתו כך | ||
| + | |- | ||
| + | | | ||
| + | ::Meaning 6 pešiṭim and 36 parts of which 154 are a whole pašuṭ. | ||
| + | ::<math>\scriptstyle{\color{blue}{a_4=\frac{24\sdot40}{154}=6+\frac{36}{154}}}</math> | ||
| + | |style="text-align:right;"|פי' ו' פשי' ל"ו חלקי' שקנ"ד פשי' שלם | ||
| + | |- | ||
| + | | | ||
| + | ::Sum 10, 15, 7, and 6 together; they are 38. Sum the parts beneath them together; they are 308, meaning 2 times 154, which is 2 pešuṭim. | ||
| + | |style="text-align:right;"|צרוף יחד י' וט"ו וז' וו' הרי ל"ח‫<ref>marg. הפוך דף א' לפניך</ref> ‫<ref>126r</ref>צרוף יחד החלקי' שתחתיהן ויעלו ח' וג' מאות דהיינו ב"פ קנ"ד וזהו ב' פשי' וד"ק | ||
| + | |||
| + | |- | ||
| + | |colspan="2"| | ||
| + | ::<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle a_1+a_2+a_3+a_4&\scriptstyle=\left(10+\frac{60}{154}\right)+\left(15+\frac{90}{154}\right)+\left(7+\frac{122}{154}\right)+\left(6+\frac{36}{154}\right)\\&\scriptstyle=\left(10+15+7+6\right)+\left(\frac{60}{154}+\frac{90}{154}+\frac{122}{154}+\frac{36}{154}\right)\\&\scriptstyle=38+\frac{308}{154}=38+\frac{2\sdot154}{154}=38+2=40\\\end{align}}}</math> | ||
| + | |- | ||
| + | !<span style=color:Green>Sons</span> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:sons|652|ew5v}}23) Riddle: a rich man talking to his sons, divided his assets and said to one of his sons: take from my purse one zahuv first, and then take a tithe of the remaining. | ||
| + | :To the second he said: take two zehuvim and a tithe of the remaining. | ||
| + | :To the third he said: take three zehuvim and a tithe of the remaining. | ||
| + | :And so on to all, first one zahuv more than the preceding should be taken, and then a tithe. | ||
| + | :How much is the money and how many are the sons, so that when they took according to their father's instruction each received as the other? | ||
| + | :<math>\scriptstyle1+\left[\frac{1}{10}\sdot\left(X-1\right)\right]=2+\frac{1}{10}\sdot\left[X-\left[\left[1+\left[\frac{1}{10}\sdot\left(X-1\right)\right]\right]+2\right]\right]</math> | ||
| + | |style="text-align:right;"|<big>כ"ג חידה</big> אדם עשיר המחלק נכסיו על פה ואומר לאחד מבניו טול מכיסי זהב בראש ואח"כ טול המעשר מן הנותרי‫'<br> | ||
| + | לשני אמר טול אתה ב' זהו' ועישור נכסי' מן הנותרי‫'<br> | ||
| + | לשלישי אמר טול אתה ג' זהו' ועישור נכסי' מן הנותרי‫'<br> | ||
| + | וכן לכולם הוסיף ליקח זהב יותר בראש מן הקדמון ואח"כ עישור<br> | ||
| + | חוד כמה המעו' כמה הבני' וכשנטלו כאשר ציום אביהם הגיע לזה כזה{{#annotend:ew5v}} | ||
| + | |- | ||
| + | | | ||
| + | ::Say: since the fraction here is a tenth, subtract 1 from ten; 9 remains. So, the number of sons is 9. | ||
| + | ::<math>\scriptstyle{\color{blue}{10-1=9}}</math> | ||
| + | |style="text-align:right;"|<big>תשובה</big> אמור לפי שהחשבון השבור שבזה הוא עשירי {{#annot:term|181,1364|dEcZ}}תפחת{{#annotend:dEcZ}} א' מעשר' ישארו ט' הרי שט' היו הבני‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Then, say: 9 times 9; the result is 81. So, the number of zehuvim is 81. | ||
| + | ::<math>\scriptstyle{\color{blue}{9\sdot9=81}}</math> | ||
| + | |style="text-align:right;"|אמור אחר זה ט'פ"ט ויעלה פ"א הרי שפ"א היו הזהובי‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Each received 9 zehuvim. | ||
| + | |style="text-align:right;"|ולכל אחד הגיע ט' זהו‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Always take one less than [the denominator of] the fraction he told his sons to take and do as I instructed you. | ||
| + | |style="text-align:right;"|וכן לעולם היאך שהיה <s>ש</s> השבור' שאמר לבניו ליקח קח אחת פחו' ועשה כמו שציויתיך | ||
| + | |- | ||
| + | ! | ||
| + | |||
| + | ==== <span style=color:Green>Motion Problem - Pursuit</span> ==== | ||
| + | |||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:two men|657|SsOL}}24) Question: a man is walking ten miles a day. His friend is walking one mile on the first day, two miles on the second day, and so on in each day he goes on walking one mile more. In how many days will he reach the one who walks 10 miles? | ||
| + | :<math>\scriptstyle10X=\sum_{i=1}^x i</math> | ||
| + | |style="text-align:right;"|<big>כ"ד אדם</big> שמהלך בכל יו' י' מילין וחבירו מהלך ביום ראשון מיל יום שני ב' מילין וכן בכל ימים מוסיף והולך מיל בכמה ימים מגיע למהלך י' מיל{{#annotend:SsOL}} | ||
| + | |- | ||
| + | | | ||
| + | ::Answer: sum 10 with 10, the result is 20. Subtract one and 19 remain, so he will reach his friend in 19 days. | ||
| + | ::<math>\scriptstyle{\color{blue}{X=\left(10+10\right)-1=20-1=19}}</math> | ||
| + | |style="text-align:right;"|<big>תשובה</big> חבר י' עם י' ויהיה כ' הסר ממנו אחת וישאר י"ט הרי שבי"ט ימים מגיע [לחבירו‫] | ||
| + | |- | ||
| + | | | ||
| + | :Thus, always double the walk of the one who walks in a constant [velocity], then subtract 1 and this is [the number of] days within which he will reach him. | ||
| + | :<math>\scriptstyle n\sdot X=\sum_{i=1}^x i\longrightarrow X=2n-1</math> | ||
| + | |style="text-align:right;"|וכן לעולם תכפול מהלכו של המהלך קביעיתו והסר אחת ובאילו ימי' הגיעו | ||
| + | |- | ||
| + | | | ||
| + | *If the second is walking 1; 3; 5; 7. | ||
| + | :<math>\scriptstyle10X=\sum_{i=1}^x \left(2i-1\right)</math> | ||
| + | |style="text-align:right;"|ואם השני מהלך אגה"ז | ||
| + | |- | ||
| + | | | ||
| + | ::Subtract 10 from the walk and he will reach him in 10 days. | ||
| + | |style="text-align:right;"|תחוסר מן המהלך י' וישיגו בי' ימי‫' | ||
| + | |- | ||
| + | | | ||
| + | *If he [= the second] is walking 2; 4; 6; 8. | ||
| + | :<math>\scriptstyle10X=\sum_{i=1}^x 2i</math> | ||
| + | |style="text-align:right;"|ואם הוא מהלך <s>בוד</s> בדו"ח | ||
| + | |- | ||
| + | | | ||
| + | ::He will reach him in 9 days. | ||
| + | |style="text-align:right;"|ישיגו [בט'] ימים | ||
| + | |- | ||
| + | | | ||
| + | :Here you have a sign concerning the walker 1, 2, 3, so that you will always know by a shortcut how many miles he walked. | ||
| + | |style="text-align:right;"|והא לך סימן לעולם על המהלך אב"ג שתדע בקיצור כמה מילין הלך | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== <span style=color:Green>Ordering Problem - Stock of Coins</span> ==== | ||
| + | |||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:stock of coins|628|PzvE}}25) If a man asks you: here is a stock of coins arranged in sequence: 1, 2, 3, 4, 5, 6. | ||
| + | :<math>\scriptstyle\sum_{i=1}^n i</math> | ||
| + | |style="text-align:right;"|<big>כ"ה אז</big> אם ישאלך אדם הנה צבור מעות מונחי' כאן וכולם הונחו על דרך אבגד"הו{{#annotend:PzvE}} | ||
| + | |- | ||
| + | | | ||
| + | ::See the last in the row, for instance, if the last in the row is 9: | ||
| + | |style="text-align:right;"|תבין בסוף השיטה הזאת ח' <s>כגון</s> כגון אם סוף השיטה ט‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Take the middle of this row, which is five, and say: 5 times 9 is 45, so the sum of the coins is 45. | ||
| + | ::<math>\scriptstyle{\color{blue}{\sum_{i=1}^9 i={\color{OliveGreen}{\left[\frac{1}{2}\sdot\left(9+1\right)\right]\sdot9}}=5\sdot9=45}}</math> | ||
| + | |style="text-align:right;"|אז קח אמצעי' אותה שיט' שהוא חמשה ואמור ה' פעמי' ט' הרי מ"ה וכך המעות מ"ה | ||
| + | |- | ||
| + | | | ||
| + | *If the last in the row is 10: | ||
| + | |style="text-align:right;"|ואם סוף השיט' [י'‫] | ||
| + | |- | ||
| + | | | ||
| + | ::Take one of the means of this row and say: 5 times 10 is 50. Add half the row, meaning 5; the total is 55. | ||
| + | |style="text-align:right;"|אז קח אחת מאמצעי' השיטה ואמור ה"פ י' הרי נ' ותוסיף עוד חצי שיטה דהיינו [ה'] היינו הכל נ"ה | ||
| + | |- | ||
| + | |colspan="2"| | ||
| + | ::<math>\scriptstyle{\color{blue}{\sum_{i=1}^{10} i=\left[\left(\frac{1}{2}\sdot10\right)\sdot10\right]+\left(\frac{1}{2}\sdot10\right)=\left(5\sdot10\right)+5=50+5=55}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :Apply this rule: | ||
| + | |style="text-align:right;"|נקוט האי כללא בידך | ||
| + | |- | ||
| + | | | ||
| + | :When the last of the row is even, multiply the middle of the row by the [last] of the row and add [the middle] of the row. | ||
| + | :<math>\scriptstyle n=2m\longrightarrow\sum_{k=1}^n k=\left[\left(\frac{1}{2}\sdot n\right)\sdot n\right]+\frac{1}{2}n</math> | ||
| + | |style="text-align:right;"|כשסוף השיטה בזוגות אז כפול אמצע השיטה על שיטה שלימה ותוסיף חצי שיטה | ||
| + | |- | ||
| + | | | ||
| + | :When the last of the row is odd, such as 7, 9, 11, 13, 15, multiply the middle of the row by the [last] of the row and you do not need to add anything. | ||
| + | :<math>\scriptstyle n=2m+1\longrightarrow\sum_{k=1}^n k=\left[\frac{1}{2}\sdot\left(n+1\right)\right]\sdot n</math> | ||
| + | |style="text-align:right;"|ואם סוף השיטה בפרודות כגו' ז' ט' י"א י"ג ט"ו אז כפול אמצעי' השיטה על כל השיטה ואינך צריך להוסיף <s>ב</s>דבר | ||
| + | |- | ||
| + | ! | ||
| + | |||
| + | ==== <span style=color:Green>Proportional Division - Three Men Sharing Food</span> ==== | ||
| + | |||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:three men sharing food|645|PhjF}}26) Riddle: two sat down to eat some bread. One had three loafs of bread and the second had two loafs of bread. A third came and ate with them. At the end of the meal, he went leaving five pešiṭim for the two. How should these two share the five pešiṭim? | ||
| + | :<math>\scriptstyle\left(2-\frac{5}{3}\right)X+\left(3-\frac{5}{3}\right)X=\frac{5}{3}</math> | ||
| + | |style="text-align:right;"|‫<ref>126v</ref><big>כ"ו חידה</big> שנים הסיבו לאכול לחם האחד יש לו ג' ככרות ולשני יש לו ב' ככרות ובא עוד שלישי ואכל עמהם ובגמר סעודה הלך לו והניח לפני השנים ה' פשי‫'<br> | ||
| + | חוד איך יחלקו אילו השנים הה' פשי‫'{{#annotend:PhjF}} | ||
| + | |- | ||
| + | | | ||
| + | ::Know and understand how much of the bread is due to each: you should say: a whole loaf and two-thirds. | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{5}{3}=1+\frac{2}{3}}}</math> | ||
| + | |style="text-align:right;"|<big>דע</big> והבן כמה שהגיע לכל אחד מן הלחמניות תצטרך לומר כיכר שלם ושני שלישי | ||
| + | |- | ||
| + | | | ||
| + | ::How much did the owner of 2 loafs lose? only a third. | ||
| + | ::<math>\scriptstyle{\color{blue}{2-\left(1+\frac{2}{3}\right)=\frac{1}{3}}}</math> | ||
| + | |style="text-align:right;"|כמה הפסיד בעל ב' ככרות רק שליש | ||
| + | |- | ||
| + | | | ||
| + | ::How much did the owner of 3 loafs lose? a loaf and a third. | ||
| + | ::<math>\scriptstyle{\color{blue}{3-\left(1+\frac{2}{3}\right)=1+\frac{1}{3}}}</math> | ||
| + | |style="text-align:right;"|וכמה הפסיד בעל ג' ככרות ככר ושליש | ||
| + | |- | ||
| + | | | ||
| + | ::We find that he gave 5 pešiṭim for 5-thirds: one takes four pešiṭim and the other take one pašuṭ. | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{5}{3}=\frac{1}{3}+\frac{4}{3}}}</math> | ||
| + | |style="text-align:right;"|נמצא שנתן עבור ה' שלישיות ה' פשי' זה נוטל ארבע פשי' וזה נוטל פשי‫' | ||
| + | |- | ||
| + | | | ||
| + | :The end. | ||
| + | |style="text-align:right;"|סליק | ||
| + | |- | ||
| + | | | ||
| + | ==== <span style=color:Green>Guessing Problem - Chosen Number</span> ==== | ||
| + | | | ||
| + | |- | ||
| + | !<span style=color:Green>MS München</span> | ||
| + | | | ||
| + | |- | ||
| + | |This is not the language of the book, but the language of the late R. Weysil. | ||
| + | |style="text-align:right;"|אין זה לשון הספר רק לשון מהר"ר ווייזיל ז"ל | ||
| + | |- | ||
| + | |I have found this in another book. | ||
| + | |style="text-align:right;"|זה מצאתי בספר אחר | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:chosen number|667|WoyO}}One thinks of any number he wants up to 32 and adds to it a half of what he thought for the first time. | ||
| + | |style="text-align:right;"|<big>יחשוב</big> <sup>החושב</sup> איזה סכום שירצה עד ל"ב והנשאל יתן לו חצי מחשבתו פעם ראשון{{#annotend:WoyO}} | ||
| + | |- | ||
| + | | | ||
| + | :If there is a half in it, he announces it. | ||
| + | |style="text-align:right;"|ויאמר לו אם יש בו חצי | ||
| + | |- | ||
| + | | | ||
| + | ::For instance: if he thought of 5, he adds 2 and a half to it, meaning 7 and a half. | ||
| + | ::<math>\scriptstyle{\color{blue}{5+\left(\frac{1}{2}\sdot5\right)=5+\left(2+\frac{1}{2}\right)=7+\frac{1}{2}}}</math> | ||
| + | |style="text-align:right;"|כגון אם היה מחשבתו ה' ונתן זה לו ב' וחצי דהיינו ז' וחצי | ||
| + | |- | ||
| + | | | ||
| + | ::He is told: "make the half into one whole" and the first time that there was a half in it is kept in memory. | ||
| + | |style="text-align:right;"|וכך יאמר לו עשה החצי לאחד שלם ואותו פעם ראשון אשר היה בו מחצה יתן למשמרת | ||
| + | |- | ||
| + | | | ||
| + | :If there is no half in it: | ||
| + | |style="text-align:right;"|ואם לא היה בו חצי | ||
| + | |- | ||
| + | | | ||
| + | ::For instance: if he thought of 4, meaning the half is 2. | ||
| + | |style="text-align:right;"|כגון אם היה מחשבתו ד' דהיינו החצי ב‫' | ||
| + | |- | ||
| + | | | ||
| + | :''How good and how pleasant'' [Psalms 133, 1]. | ||
| + | |style="text-align:ri''ght;"|{{#annot:Ps133-1|494|NRky}}מה טוב ומה נעים{{#annotend:NRky}}''‫<ref group=note>תהילים קלג, א</ref> | ||
| + | |- | ||
| + | | | ||
| + | :Then, he is told: "add another half to the number you have" and he is asked if there is a half in it, or not, as I wrote. | ||
| + | |style="text-align:right;"|ואח"כ יאמר לו קח עוד חצי על חשבון אשר בידך וישאל לו אם יש בו מחצה אם לא כמו שכתבתי | ||
| + | |- | ||
| + | | | ||
| + | :And so on: he adds half to the number he has five times and each time there is a half in it, it is kept in memory. | ||
| + | |style="text-align:right;"|וככה יתן לו החצי על החשבון אשר בידו חמשה פעמי' וכל פעם אשר עלה בידו מחצה יתן למשמרת | ||
| + | |- | ||
| + | | | ||
| + | :Then, he goes to the line and every time there is a half, he takes the number written there. He casts out the 32 and what he is left with is the number he thought of. | ||
| + | |style="text-align:right;"|ואח"כ ילך אל זה השורה וכל פעם אשר היה בו מחצה יקח לו אותו מספר אשר כתוב כאן וישלך אותו בל"ב ל"ב ומה שנשאר בידו הוא המספר <s>אשר</s> אשר חושב השואלו | ||
| + | |- | ||
| + | | | ||
| + | ::For instance, if there was a half in the second time, in the fourth time and in the fifth time: take what is written for the second time, i.e. the forefinger, which is 14; for the fourth time, i.e. 24; and for the fifth time, which is 16. Cast out 32; 22 remains and this is the number he was thinking of. | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(14+24+16\right)-32=22}}</math> | ||
| + | |style="text-align:right;"|כגון אם היה בפעם שנית ובפעם רביעי' ובפעם חמישי' מחצה א"ב קח מה דכתי' אצל פעם שנית דהיינו אצבע והוא ד"א <s>אל</s> ואצל פעם רביעי' דהיינו ד"ב ואצל חמישית דהוא ו"א והשלך בל"ב ל"ב וישאר כ"ב דהיינו החשבון <s>אצל</s> <sub>קל"ב</sub> אשר חשב | ||
| + | |- | ||
| + | | | ||
| + | :And so it always is. | ||
| + | |style="text-align:right;"|וכן לכל פעם | ||
| + | |- | ||
| + | | | ||
| + | *Likewise, if the one who asks wants him to think of any number up to 128 | ||
| + | |style="text-align:right;"|‫<ref>125r</ref>וכן אם ירצה השואל יחשוב חשבון עד קכ"ח | ||
| + | |- | ||
| + | | | ||
| + | :Tell him as I instructed, but [now] he has to add half the number seven times and every time there is a half there, he takes one for the line I wrote. | ||
| + | |style="text-align:right;"|והנשאל אמור לו כמה <s>אמור</s> שציויתיך רק שבעה פעמי' יתן לו חצי החשבון ומתי שהיה בו מחצה יקח אחד מן השורה אשר אכתוב | ||
| + | |- | ||
| + | | | ||
| + | ::For instance, if one thinks of a number, such that there is a half in the first time, the third time, the fifth time and the seventh: [take] 43 for the first line, 86 for the third, 48 for the fifth, and 64 for the seventh. Sum them up; it is 241. Cast out the 32s; 17 remains and this the original number he thought of. | ||
| + | ::<math>\scriptstyle{\color{blue}{43+86+48+64=241\pmod{32}17}}</math> | ||
| + | |style="text-align:right;"|כגון אם חושב השואל חשבון שבפעם ראשון ובפעם שלישי ובפעם חמישי ובפעם שביעי' היה בו מחצה דהיינו חשבונם לשורה ראשונה ג"ד ולשלישי ו"ח ולחמשי ח"ד ולשביעי ד"ו צרוף יחד ויהיה אד"ב השליכהו בל"ב ל"ב וישאר ז"א דהיינו החשבון הראשון אשר חשב השואל | ||
| + | |- | ||
| + | | | ||
| + | ::Your sign: | ||
| + | |style="text-align:right;"|סימניך | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |21||thumb||20-1 | ||
| + | |- | ||
| + | |14||forefinger||4-10 | ||
| + | |- | ||
| + | |20||middle finger||10-2-8 | ||
| + | |- | ||
| + | |24||ring finger||10-4-10 | ||
| + | |- | ||
| + | |16||little finger||4-10-2 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |א"ב||גודל||אך | ||
| + | |- | ||
| + | |ד"א||אצבע||יד | ||
| + | |- | ||
| + | |‫0"ב||אמה||חבי | ||
| + | |- | ||
| + | |ד"ב||קמיצה||ידי | ||
| + | |- | ||
| + | |ו"א||זרת||ביד | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | ! | ||
| + | |||
| + | ==== <span style=color:Green>Multiple Quantities - Men, Women, Children</span> ==== | ||
| + | |||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:men, women, children|652|zAxf}}Ask a riddle: people came to eat. Among them, there were men, women, and children. All in all 20 [people]. The host came and said to them: every man should give me three pešiṭim, every woman – two pešiṭim, and every child – a half pašuṭ, and so they did. The total amount of money [paid by] all of them together was 20. | ||
| + | :Ask: how many men, women, and children were there? | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle3X+2Y+\frac{1}{2}Z=20\\\scriptstyle X+Y+Z=20\end{cases}</math> | ||
| + | |style="text-align:right;"|<big>חוד</big> חידה הנה באו הנה נפשות לאכול ויש ביניהם אנשים ונשים וטף וסך כולם עשרים ובא להן בעל אושפיזן ואמר להן תנו לי איש אחד מכם ג' פשי' ואשה ב' פשי' ותינוק <sup>חצי</sup> פשי' וכן עשו ויעלה הסך של מעות כולם עשרים פשי‫'<br> | ||
| + | חוד כמה היו האנשים וכמה הנשים וכמה הטף{{#annotend:zAxf}} | ||
| + | |- | ||
| + | | | ||
| + | ::Answer: one man, five women, and 14 children. | ||
| + | |style="text-align:right;"|תשוב איש אחד חמשה נשים י"ד תינוקות | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{\begin{cases}\scriptstyle men=X=1\\\scriptstyle women=Y=5\\\scriptstyle children=Z=14\end{cases}}}</math> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :Note well. | ||
| + | |style="text-align:right;"|ודוק | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:men, women, children|652|jTPd}}Another way: a hundred people came to a guesthouse and sat to eat together. Among them, there were men, women, and children. The host came and said to them: every man should give me ten pešiṭim, every woman – five pešiṭim, and every child – a half pašuṭ, and so they did. The total amount of money [paid by] all of them together was 100 pešiṭim. | ||
| + | :Ask: how many men, women, and children were there? | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle10X+5Y+\frac{1}{2}Z=100\\\scriptstyle X+Y+Z=100\end{cases}</math> | ||
| + | |style="text-align:right;"|<big>דרך</big> אחר הנה באו מאה נפשות למלון אחד וישבו יחד לאכול ויהיה ג"כ ביניהם [אנשים]‫<ref>M: אשנים</ref> [ונשים]‫<ref>M: ושנים</ref> וטף ובא ג"כ להן בעל אושפיזין ואמר להן תנו לי כל איש עשרה פשי' ואשה ה' פשי' ותינוק חצי פשי' וכן עשו ויעלה הסך של מעות מאה פשי‫'<br> | ||
| + | חוד כמה אנשים וכמה הנשי' וכמה תינוקות{{#annotend:jTPd}} | ||
| + | |- | ||
| + | | | ||
| + | ::Answer: one man, nine women, and ninety children. | ||
| + | |style="text-align:right;"|תשובה איש אחד ותשעה נשים ותשעים תינוקו‫' | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{\begin{cases}\scriptstyle men=X=1\\\scriptstyle women=Y=9\\\scriptstyle children=Z=90\end{cases}}}</math> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | ==== <span style=color:Green>Double False Position <br>Find a Quantity Problem - How Much Problem - Money</span> ==== | ||
| + | |||
| + | | | ||
| + | |- | ||
| + | |Question by R. Israel from Prague: | ||
| + | |style="text-align:right;"|שאלה מפי הר"ר ישראל מפראג | ||
| + | |- | ||
| + | |A number that we add to it, how much was it originally? | ||
| + | |style="text-align:right;"|ממון שהוספנו עלינו כמה שהיה בתחלה | ||
| + | |- | ||
| + | | | ||
| + | :For instance, if it is 3; you add 3 to it, as well as its half and its quarter; the total is twenty, or 30, or 40, or as much as you wish. | ||
| + | |style="text-align:right;"|כגון אם הוא ג' תוסיף עליו ג' וגם מחציתו ורביעתו ויהיה הכל עשרים או ל' או מ' או כפי רצונך כמה שתרצה | ||
| + | |- | ||
| + | |Write two false numbers and use them to calculate the truth, how much it was originally. | ||
| + | |style="text-align:right;"|ותכתוב שני חשבונות שקרים ותחשוב מהם האמת כמה היה בתחילה | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:money|648|kDrg}}An amount of money, we add to it as it was at first, plus its half and its quarter and the total is 20. | ||
| + | :<math>\scriptstyle X+X+\frac{1}{2}X+\frac{1}{4}X=20</math> | ||
| + | |style="text-align:right;"|והנה לך דימיון כגון ממון שהוספנו עליו כמו שהיה בתחילה ומחציתו ורביעתו ויהיה הכל עשרים{{#annotend:kDrg}} | ||
| + | |- | ||
| + | | | ||
| + | ::Think as if the amount of money was originally 4: we add another 4 to it, as well as its half, which is 2, and its quarter, which is 1; the total is 11. | ||
| + | ::'''<span style=color:Green>(1)</span>''' <math>\scriptstyle{\color{blue}{4+4+\left(\frac{1}{2}\sdot4\right)+\left(\frac{1}{4}\sdot4\right)=4+4+2+1=11}}</math> | ||
| + | |style="text-align:right;"|אז חשוב אם היה בתחלה המעות ד' זהו' והוספנו עליו עוד ד' ועוד מחציתו שהוא ב' ורביעתו שהוא א' והנה הכל ביחד א'א‫' | ||
| + | |- | ||
| + | | | ||
| + | ::So, this is false, because the total should be 20, as we required that when we add the same to it, plus its half and its quarter, the total will be twenty; and this is only 11. | ||
| + | |style="text-align:right;"|והנה הוא שקר כי היה ראוי להיות הכל 0"ב כי שאילתינו כשהוספנו עליו כמו שהיה בתחילה ומחציתו ורביעתו ויהיה הכל עשרים וזה אינו רק א'א‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Think how much it is from 11 to 20; it is 9. | ||
| + | ::<math>\scriptstyle{\color{blue}{20-11=9}}</math> | ||
| + | |style="text-align:right;"|אז חשוב כמה הוא מן א'א' עד 0"ב ויהיה ט‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Write 9 beneath the 4 we said the amount of money was originally; like this: | ||
| + | |style="text-align:right;"|אותו ט' כתוב תחת ד' שאמרנו שהיו המעות בתחילה כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color: blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |4 | ||
| + | |- | ||
| + | |9 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ד | ||
| + | |- | ||
| + | |ט | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Then, think as if the amount of money was originally 8: we add another 8 to it, according to our question, as well as its half, which is 4, and its quarter, which is 2; the total is 22. | ||
| + | ::'''<span style=color:Green>(2)</span>''' <math>\scriptstyle{\color{blue}{8+8+\left(\frac{1}{2}\sdot8\right)+\left(\frac{1}{4}\sdot8\right)=8+8+4+2=22}}</math> | ||
| + | |style="text-align:right;"|ואח"כ חשוב אם היו המעו' בתחילה ח' והוספנו עליו כפי שאילתינו עוד ח' ומחציתו שהוא ד' ורביעיתו שהוא ב' והנה הכל ביחד ב'ב‫' | ||
| + | |- | ||
| + | | | ||
| + | ::So, this is also false, because the total should be 20, as we required. For we required that when we add the same to it, plus its half and its quarter, the total will be 20. | ||
| + | |style="text-align:right;"|א"כ זהו ג"כ שקר כי הוא ראוי להיות הכל ביחד 0"ב כמו ששאלנו כי שאלנו כשהוספנו עליו כמו שהיה בתחילה ומחציתו ורביעתו ויהיה הכל 0"ב | ||
| + | |- | ||
| + | | | ||
| + | ::Subtract 20 from the number; it exceeds by 2. | ||
| + | ::<math>\scriptstyle{\color{blue}{22-20=2}}</math> | ||
| + | |style="text-align:right;"|אז תנכה 0"ב מן החשבון כפי שאילתנו ויהיה יותר ב‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Write 2 beneath the 8 we said the amount of money was originally. | ||
| + | |style="text-align:right;"|אותו ב' כתוב תחת ח' שאמרנו שהיה המעות בתחילה | ||
| + | |- | ||
| + | | | ||
| + | ::Write 8 to the left of 4 and write the 2 that remains from this number to the left of 9; like this: | ||
| + | |style="text-align:right;"|וכתוב ח' בצד שמאל של ד' וגם ב' הנשאר מן החשבון כתוב בצד שמאל על ט' כזה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color: blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |8||4 | ||
| + | |- | ||
| + | |2||9 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ח||ד | ||
| + | |- | ||
| + | |ב||ט | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Add 2 and 9 together, because they are the remainders of the false numbers; it is 11 and this is the denominator. | ||
| + | |style="text-align:right;"|והנה צרוף ט"ב ביחד כי הם נשארים מן החשבונות השקרים ויהיה א'א' וזה המורה | ||
| + | |- | ||
| + | | | ||
| + | ::Then, multiply the 2 beneath [the 8] by 4; it is 8. | ||
| + | |style="text-align:right;"|אח"כ כפל ב' שתחת על ד' ויהיה ח‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Write 8, so you will not forget. | ||
| + | |style="text-align:right;"|אותו ח' כתוב שלא תשכח | ||
| + | |- | ||
| + | | | ||
| + | ::Next, multiply the 9 beneath the 4 by 8; it is 72. | ||
| + | |style="text-align:right;"|ואח"כ כפול ט' שתחת ד' על ח' ויהיה ב"ז | ||
| + | |- | ||
| + | | | ||
| + | ::Take the 8 you already wrote and add it to 2; it is ten. | ||
| + | |style="text-align:right;"|וקח ח' שכתבת כבר ושים אותו על ב' ויהיה עשרה | ||
| + | |- | ||
| + | | | ||
| + | ::Write zero instead of the 2; erase the 4 and write 8 instead; it is 80. | ||
| + | |style="text-align:right;"|כתוב גלגל במקום ב' ומחוק ד' וכתוב במקומה ח' ויהיה 0"ח פי' פ‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Then, divide according to the division chapter: how many times is 11 in eighty? Because this is the denominator. You find it 7 times and 3 parts of 11 of a whole zahuv and this is the original amount of money we asked for. The one who understands will understand. | ||
| + | ::<math>\scriptstyle{\color{blue}{x=\frac{\left(2\sdot4\right)+\left(9\sdot8\right)}{9+2}=\frac{8+72}{11}=\frac{80}{11}=7+\frac{3}{11}}}</math> | ||
| + | |style="text-align:right;"|ואח"כ חלוק בשער החילוק כמה פעמים א'א' יש בשמונים כי הוא המורה ותמצא בו ז' פעמים ועוד ג' חלקים שא'א' מהן חלק שלם פי' שלם זהב שלם וכך היא המעות בתחילה ששאלנו והמבין יבין | ||
| + | |- | ||
| + | |The above calculation is correct when one of the two false numbers is deficient and the other is exceeding. | ||
| + | |style="text-align:right;"|וזה החשבון דלעיל מכוון כששני חשבונות השקרים אחד פחות ואחד יותר | ||
| + | |- | ||
| + | |But, if the two false numbers both exceed the [original] number, or both deficient, I will teach you what to do then: | ||
| + | |style="text-align:right;"|אבל אם שני חשבונות שקרים כל אחד יותר על חשבון היוצא או שניהם חסרים אשכילך האיך תעשה אז | ||
| + | |- | ||
| + | |Subtract the smaller remainder from the greater remainder and what you are left with is the denominator. | ||
| + | |style="text-align:right;"|תנכה החשבון המועט הנשאר מן חשבון המרובע ומה שישאר לך זהו המורה | ||
| + | |- | ||
| + | |When multiplying the two numbers by each other, subtract also the smaller number from the greater. | ||
| + | |style="text-align:right;"|וכשכפלת שני חשבונות יחד אז תנכה גם כן חשבון המועט מן המרובה | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:money|648|nH9r}}I shall write you an example, for instance, if you have an amount of money, you add to it the same amount, and its half and its quarter and the total is 20 | ||
| + | :<math>\scriptstyle X+X+\frac{1}{2}X+\frac{1}{4}X=20</math> | ||
| + | |style="text-align:right;"|והנה אכתוב לך דימיון כגון אם יש לך סך מעות '''וכפלת עליו''' סך שהיה כבר ומחציתו ורביעתו ויהיה בין הכל 0"ב{{#annotend:nH9r}} | ||
| + | |- | ||
| + | | | ||
| + | ::I will teach you how to operate: | ||
| + | |style="text-align:right;"|אשכילך האיך תעשה | ||
| + | |- | ||
| + | | | ||
| + | ::If you say that the amount of money was 8 zehuvim: we add another 8 to it, as well as its half, which is 4, and its quarter, which is 2; the total is 22. | ||
| + | ::'''<span style=color:Green>(1)</span>''' <math>\scriptstyle{\color{blue}{8+8+\left(\frac{1}{2}\sdot8\right)+\left(\frac{1}{4}\sdot8\right)=8+8+4+2=22}}</math> | ||
| + | |style="text-align:right;"|אם תאמר שהיה סך מעות ח' זהו' והוספנו עליו עוד ח' ומחציתו שהוא ד' ורביעתו שהוא ב' ויהיה [ס]ך הכל ב'ב‫' | ||
| + | |- | ||
| + | | | ||
| + | ::This is false, because the total should be 20. | ||
| + | |style="text-align:right;"|וזה שקר כי היה ראוי להיות סך הכל 0"ב | ||
| + | |- | ||
| + | | | ||
| + | ::Subtract 20; 2 remains. | ||
| + | ::<math>\scriptstyle{\color{blue}{22-20=2}}</math> | ||
| + | |style="text-align:right;"|אז תנכה 0"ב וישאר ב‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Write 2, so you will not forget. | ||
| + | |style="text-align:right;"|אותו ב' כתוב שלא [ת]שכח | ||
| + | |- | ||
| + | | | ||
| + | ::Then, think about the second number and say: if the amount of money was 16: we add 16 to it, as well as its half, which is 8, and its quarter, which is 4; the total is 44. | ||
| + | ::'''<span style=color:Green>(2)</span>''' <math>\scriptstyle{\color{blue}{16+16+\left(\frac{1}{2}\sdot16\right)+\left(\frac{1}{4}\sdot16\right)=16+16+8+4=44}}</math> | ||
| + | |style="text-align:right;"|ואז תחשוב חשבון השני ותאמר אם היו סך המעות ו"א והוספנו עליו ו"א ומחציתו שהוא ח' ורביעיתו שהוא ד' ויהיה בין הכל ד"ד | ||
| + | |- | ||
| + | | | ||
| + | ::This is a complete false, because it should be 20. | ||
| + | |style="text-align:right;"|וזה שקר גמור כי היה ראוי להיות 0"ב | ||
| + | |- | ||
| + | | | ||
| + | ::So, subtract 20, which is the resulting total, from 44; 22 remains. | ||
| + | ::<math>\scriptstyle{\color{blue}{44-20=24}}</math> | ||
| + | |style="text-align:right;"|אז תנכה 0"ב שהוא סך היוצא מן מעות מן ד"ד וישאר ד"ב | ||
| + | |- | ||
| + | | | ||
| + | ::Subtract 2, which is the remainder of the first number, from 24, which is the remainder of the second number; 22 remains and this is the denominator. | ||
| + | |style="text-align:right;"|ואח"כ ב' הנשאר מן חשבון הראשון מן ד"ב הנשאר מן חשבון השני וישאר ב"ב וזהו המורה | ||
| + | |- | ||
| + | | | ||
| + | ::Then, multiply the 2 beneath the 8 by 16; it is 32. Write it, so you will not forget. | ||
| + | |style="text-align:right;"|ואז כפול ב' שתחת ח' על ו"א ויהיה ב"ג כתוב שלא תשכח | ||
| + | |- | ||
| + | | | ||
| + | ::Next, multiply the 24 by 8; it is 192. | ||
| + | |style="text-align:right;"|ואח"כ כפול ד'ב' על ח' ויעלה ב'ט'א‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Subtract the first product, which is 32, from the second [product], which is 192, because it is the smaller number; 160 remains. | ||
| + | |style="text-align:right;"|אז תנכה החשבון הראשון אשר כפלת שהוא ב"ג מן החשבון השני שהוא ב'ט'א' כי הוא חשבון המועט וישאר 0'ו'א‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Then, divide according to the division chapter: how many times is 22 in it? Because this is the denominator. You find it 7 times and 6 parts of 22 of a whole unit, i.e. zahuv. The one who understands will understand. | ||
| + | ::<math>\scriptstyle{\color{blue}{x=\frac{\left(24\sdot8\right)+\left(2\sdot16\right)}{24-2}=\frac{192-32}{22}=\frac{160}{22}=7+\frac{6}{22}}}</math> | ||
| + | |style="text-align:right;"|אז חלוק בשער החילוק כמה פעמים ב'ב' יש בו כי הוא המורה ותמצא שיש בו ז' פעמים וגם ו' חלקים שב'ב' מהן חלק שלם דהיינו זהו' והמבין יבין סליק | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | === <span style=color:Green>Additional Word Problems</span> === | ||
| + | |||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | ==== <span style=color:Green>Mantova, Comunità Ebraica MS ebr. 8/16</span> ==== | ||
| + | | | ||
| + | |- | ||
| + | |I will reveal to you the chapter on fractions: | ||
| + | |style="text-align:right;"|והנה אגלה לך שער השברים | ||
| + | |- | ||
| + | | | ||
| + | *If one asks you about a piece [of bread]: into how many parts you need to divide the piece [of bread], so that you find a third, a quarter, and a fifth of it? | ||
| + | |style="text-align:right;"|אם נשאל לך השואל על פת אחת לכמה חלקים שצריך אתה לשבר הפת שתמצא בה שלישית ורביעית וחמישית | ||
| + | |- | ||
| + | | | ||
| + | :Write the question as follows: | ||
| + | |style="text-align:right;"|אז תכתוב כך השאלה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color: blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |5 | ||
| + | |- | ||
| + | |4 | ||
| + | |- | ||
| + | |3 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |style="text-align:right;"|ה | ||
| + | |- | ||
| + | |style="text-align:right;"|ד | ||
| + | |- | ||
| + | |style="text-align:right;"|ג | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Say: 3 times 4; the result is 12. | ||
| + | |style="text-align:right;"|ותאמ' ג' פעמים ד' ויעלה ב"א | ||
| + | |- | ||
| + | | | ||
| + | ::Multiply the 12 once by 5; you receive 60 and these are the parts. | ||
| + | ::<math>\scriptstyle{\color{blue}{3\times4\times5=12\times5=60}}</math> | ||
| + | |style="text-align:right;"|ותכפול פעם אחת הב"א תוך הה' אז יעל' לך 0"ו וזה חלוקים | ||
| + | |- | ||
| + | | | ||
| + | ::You find the third, quarter and fifth: 20 is a third of 60; the quarter is 15 and the fifth is 12. | ||
| + | |style="text-align:right;"|שתמצא שלישית ורביעית וחמישית דהא 0"ב שלישית מ0"ו ורביעית ה"א וחמישית זהו ב"א סליק | ||
| + | |- | ||
| + | |colspan="2" | | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{1}{3}\sdot60=20\quad\frac{1}{4}\sdot60=15\quad\frac{1}{5}\sdot12}}</math> | ||
| + | |- | ||
| + | |I will reveal to you the chapter on ratio and this its example: | ||
| + | |style="text-align:right;"|והנה אגלה לך שער הערך וזהוא דמיונו | ||
| + | |- | ||
| + | | | ||
| + | *If one asks you: you have 12 barrels that are bought for 6 zehuvim, how much are 9 barrels cost? | ||
| + | |style="text-align:right;"|אם נשאל לך השואל הנה לפניך ב"א חביות שנקנין עבור ו' זהובי‫'<br> | ||
| + | ט' חביות כמה הן עולין | ||
| + | |- | ||
| + | | | ||
| + | :Write the question as follows: | ||
| + | |style="text-align:right;"|אז תכתו' כך השאלה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color: blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |9 | ||
| + | |- | ||
| + | |6 | ||
| + | |- | ||
| + | |12 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |style="text-align:right;"|ט | ||
| + | |- | ||
| + | |style="text-align:right;"|ו | ||
| + | |- | ||
| + | |style="text-align:right;"|בא | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Say: 6 times 9; you receive 54. | ||
| + | |style="text-align:right;"|ואז תאמר ו' פעמי' ט' ויעלה לך ד"ה | ||
| + | |- | ||
| + | | | ||
| + | ::Divide 54 by 12: | ||
| + | |style="text-align:right;"|אז תחלוק הב"א מד"ה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color: blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |54 | ||
| + | |- | ||
| + | |12 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |style="text-align:right;"|דה | ||
| + | |- | ||
| + | |style="text-align:right;"|בא | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Say: how many times is 1 in 5? 1 is 5 times in 5. | ||
| + | |style="text-align:right;"|ותאמר כמה א' בה' והא' הוי תוך הה' ה' פעמים | ||
| + | |- | ||
| + | | | ||
| + | ::But, you cannot take 5 times, because you will not be able to take the 2 five times from 4 and as the number of times you take the 1 from the 5, so is the number of times you have to take the 2 from 4. Therefore, you can take the 1 only 4 times from the 5 and then you have 14 left. | ||
| + | |style="text-align:right;"|אבל לא תוכל ליקח ה' פעמים דאל"כ לא תוכל ליקח הב' מן הד' ה' פעמים וכמה פעמים שתקח הא' מן הה' כך הרבה פעמים צריך אתה ליקח הב' מן הד' אלא לא תוכל ליקח הא' מן הה' אלא ד' פעמים ואז נשאר לך ד"א | ||
| + | |- | ||
| + | | | ||
| + | ::Write the 4 above. | ||
| + | |style="text-align:right;"|והד' תכתו' למעלה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color: blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |4 | ||
| + | |- | ||
| + | |12 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |style="text-align:right;"|ד | ||
| + | |- | ||
| + | |style="text-align:right;"|בא | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::The 4 are the zehuvim. | ||
| + | |style="text-align:right;"|דה"ד הוי הזהו‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Then, you should take the 2 also 4 times from 14; you are left with 6 and these are the greats [= grossi], of which 12 are one zahuv. | ||
| + | |style="text-align:right;"|ואז צריך ליקח הב' מן ד"א נמי ד' פעמי' אז נשאר לך ו' וזהו ג' גדולי' שעולי' י"ב על הזהוב אחד | ||
| + | |- | ||
| + | | | ||
| + | ::We find that when you buy 12 barrels for 6 zehuvim, 9 barrels are cost 4 zehuvim and a half. | ||
| + | |style="text-align:right;"|ונמצא לכשתקנה ב"א חביות עבור ו' זהובי' שט' חביות עולים ד' זהובי' וחצי | ||
| + | |- | ||
| + | | | ||
| + | :Do this every time. | ||
| + | |style="text-align:right;"|כן תעשה לכל פעם | ||
| + | |- | ||
| + | |I will explain to you another ratio: | ||
| + | |style="text-align:right;"|ועוד אפרש לך ערך אחר | ||
| + | |- | ||
| + | | | ||
| + | *Two lent a loan of 34 zehuvim - one had a debt of 15 zehuvim and the other had a debt of 19 zehuvim and they charged an interest of 13 zehuvim. | ||
| + | :How much will each have of the 13 zehuvim according to this ratio? | ||
| + | |style="text-align:right;"|שנים שהלוו על חוב אחד ד"ג זהובי' ולאחד יש לו בחוב ה"א זהובי' ולשיני יש לו בחוב ט"א זהובי' ולקחו שנים לרבית ג"א זהובי‫'<br> | ||
| + | כמה יש לכל אחד בה ג"א זהובי' לפי הערך | ||
| + | |- | ||
| + | | | ||
| + | :I will briefly explain to you how to operate, so that you will find it right: | ||
| + | |style="text-align:right;"|והנה אפרש לך בקיצור איך תעשה שתמצא היושר | ||
| + | |- | ||
| + | | | ||
| + | ::Write each one separately and this is how you write: | ||
| + | |style="text-align:right;"|אז תכתו' כל אחד לחוד וכך תכתו‫' | ||
| + | |- | ||
| + | | | ||
| + | ::The interest is above, then the money he has in debt, and then the whole amount. | ||
| + | |style="text-align:right;"|הריבי' למעלה ואח"כ המעות שיש לו בחוב ואח"כ הסך כולה | ||
| + | |- | ||
| + | | | ||
| + | ::This is the diagram that is written in the margin: | ||
| + | |style="text-align:right;"|וזה דומיונו שכתו' מבראי | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color: blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | | ||1||3 | ||
| + | |- | ||
| + | |1||5|| | ||
| + | |- | ||
| + | | ||3||4 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |style="text-align:right;"| ||א||ג | ||
| + | |- | ||
| + | |style="text-align:right;"|א||ה|| | ||
| + | |- | ||
| + | |style="text-align:right;"| ||ג||ד | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Multiply his money by the interest and say: 1 times 1; then say: 1 times 5, which is 5. | ||
| + | |style="text-align:right;"|ותכפל המעות שלו תוך הריבית ותאמ' פ' א'פ'א' ואח"כ תאמ' א'פ'ה' שהוא ה‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Write 15 again beneath the upper 3. The diagram is like this: | ||
| + | |style="text-align:right;"|ואח"כ תכתו' עוד פעם אחת ה"א תחת הג' שלמעלה ויהיה כך דמיונו | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color: blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |1||5||3 | ||
| + | |- | ||
| + | | ||1||5 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |א||ה||ג | ||
| + | |- | ||
| + | | ||א||ה | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Say: 3 times 1 is 3. Add it to the upper 5; it is 8. | ||
| + | |style="text-align:right;"|ותאמ' גפ"א שהוא ג' ותשים על הה' שלמעלה אז יהיה ח‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Again say: 3 times 5 is 15. | ||
| + | |style="text-align:right;"|ותאמ' עוד פ"א גפ"ה שהוא ה"א | ||
| + | |- | ||
| + | | | ||
| + | ::You find it is 195. | ||
| + | |style="text-align:right;"|אז תמצא ה'ט'א‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Divide 195 by 34 and say: how many 3s are there in 19? It is 6 times in it. | ||
| + | |style="text-align:right;"|אז תחליק הד"ג מן הט"א ותאמ' כמה יש ג' בתוך ט"א והוא הוי ביה ו'פ‫' | ||
| + | |- | ||
| + | | | ||
| + | ::But, you cannot take it 6 times, because then you will only have 15 left and you cannot take 6 times 4 from 15. | ||
| + | |style="text-align:right;"|אבל תוכל לקח ו' פ' כי אז אין נשאר לך אלא ה'א' ולא תוכל ליקח ו'פ'ד' מה"א | ||
| + | |- | ||
| + | | | ||
| + | ::You can only take 5 times, so you have 45 left. | ||
| + | |style="text-align:right;"|אלא לא תוכל ליקח אל ה'פ' אז נשאר לך הד‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Write the 5 above. | ||
| + | |style="text-align:right;"|והה' תכתו' למעלה כמו כן | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color: blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | | ||5 | ||
| + | |- | ||
| + | |4||5 | ||
| + | |- | ||
| + | | ||4 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | | ||ה | ||
| + | |- | ||
| + | |ד||ה | ||
| + | |- | ||
| + | | ||ד | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Take 5 times 4 from 45; you are left with 25. | ||
| + | |style="text-align:right;"|ותקח ה'פ'ד' מן ה'ד' אז תשאר לך ה"ב | ||
| + | |- | ||
| + | | | ||
| + | ::Hence, his share is 5 zehuvim and 2 greats, of which 34 are one zahuv. | ||
| + | |style="text-align:right;"|אז יהיה לזה בחלקו ה' זהוב' והב' גדולי' שעולים ד"ג על זהוב אחד | ||
| + | |- | ||
| + | | | ||
| + | ::You do the same for the other and you find that the share of the other is 7 zehuvim and 9 greats, of which 34 are one zahuv. | ||
| + | |style="text-align:right;"|ועשית' גם כן לצד השני ותמצא שיהיה לשני בחלקו ז' זהובי' וט' גדולי' שעולי' ד"ג על זהוב אחד סליק | ||
| + | |- | ||
| + | !<span style=color:Green>Boiling Water Problem</span> | ||
| + | | | ||
| + | |- | ||
| + | |I will explain to you another ratio: | ||
| + | |style="text-align:right;"|הנה אפרש לך ערך אחר | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:WP|663|f9hE}}If one asks you: You have 15 se'ah of water, if they are boiled on fire for one day, 7 se'ah are evaporated. | ||
| + | :If there are only 13 se'ah on fire, how much will be evaporated in one day according to this ratio? | ||
| + | :<math>\scriptstyle\frac{15}{7}=\frac{13}{X}</math> | ||
| + | |style="text-align:right;"|אם ישאל לך השואל כי הנה ה"א סאין מים לפניך ואם מתבשלין על האור יום אחד אז הוי מתמעטין ז' סאין<br> | ||
| + | הנה אם היו רק ג"א סאין על האש והאש הוי מממועט לפי זה הערך כמה הוי מתמעט מן הג"א סאין ביום אחד{{#annotend:f9hE}} | ||
| + | |- | ||
| + | | | ||
| + | ::Write the question as written in the margin: | ||
| + | |style="text-align:right;"|אז תכתו' כך השאלה כדכתי' מבדאי | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color: blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | | ||7 | ||
| + | |- | ||
| + | |1||3 | ||
| + | |- | ||
| + | |1||5 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | | ||ז | ||
| + | |- | ||
| + | |א||ג | ||
| + | |- | ||
| + | |א||ה | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Do as I explained above: multiply the 13 by the 7; you find 91. | ||
| + | |style="text-align:right;"|ותעשה כמו שפרשתי לעיל תכפול הג"א תוך הז' ותמצא א"ט | ||
| + | |- | ||
| + | | | ||
| + | ::Then, divide 91 by 15; you find that 6 se'ah and one part of 15 of one se'ah evaporate in one day out of the 13 se'ah. | ||
| + | ::<math>\scriptstyle{\color{blue}{X=\frac{13\times7}{15}=\frac{91}{15}=6+\frac{1}{15}}}</math> | ||
| + | |style="text-align:right;"|אז תחלוק ה"א מן הא"ט ותמצא שהוי הג"א סאין מתמעט ביום אחד ו' סאין וחלק אחד שה"א עושין על סאה אחת סליק | ||
| + | |- | ||
| + | |The [chapter] on ratio | ||
| + | |style="text-align:right;"|השורש מערך | ||
| + | |- | ||
| + | | | ||
| + | *Two lent a loan of 9 zehuvim - one has 5 zehuvim and the other [has] 4 zehuvim and they charged an interest of 13 [zehuvim]. | ||
| + | |style="text-align:right;"|שנים שהלוו על חוב ט' זהו' לאחד יש ה' זהו' ולאחד ד' זהו' ולקחו לרבית י"ג | ||
| + | |- | ||
| + | | | ||
| + | ::We find that each has an interest of 13-ninths of one zahuv. | ||
| + | |style="text-align:right;"|ונמצא דשייך לכל אחד בזהוב י"ג תשיעיו' לרבית | ||
| + | |- | ||
| + | | | ||
| + | ::We find that the one who has 5 zehuvim has 5 times 13-ninths, which are [65]-ninths, when you divide it by 9, which is the denominator, meaning each 9-ninths are an interest of one zahuv. | ||
| + | |style="text-align:right;"|ונמצא דשייך לזה שיש לו ה' זהו' ה'פ' י"ג תשיעיו' שהם וו' תשיעיו' וכשתחלוק ממנו ט' שהוא המור' פי' כל ט' תשיעיות הם רבי' זהוב | ||
| + | |- | ||
| + | | | ||
| + | ::Do the same for the other. | ||
| + | |style="text-align:right;"|וכך תעשה גם לשני סליק | ||
| + | |- | ||
| + | |I will explain to you a ratio of a ratio: | ||
| + | |style="text-align:right;"|והנה אפרש לך ערך מתוך ערך | ||
| + | |- | ||
| + | | | ||
| + | *One lent a loan of 9 zehuvim for 14 weeks, another lent a loan of 11 zehuvim for 15 weeks and they charged together an interest of 7 zehuvim. | ||
| + | :How much will each have according to this ratio? | ||
| + | |style="text-align:right;"|והנה אחד שלוה על חוב ט' זהובי' והם עומדים ד"א שבועות ואחד שלוה על חוב א"א זהובי' והם עומדי' ה"א שבועות ולקחו שניהם ליחד ריבית ז' זהובי‫'<br> | ||
| + | כמה יש לכל אחד בחלקו לפי הערך | ||
| + | |- | ||
| + | | | ||
| + | ::I will explain to you how to operate in order to find the truth: | ||
| + | |style="text-align:right;"|הנה אפרש לך בקיצור איך שתעשה שתמצ' האמת | ||
| + | |- | ||
| + | | | ||
| + | ::Write for each one separately as it is written in the margin: the interest above, then the amount of money and then the [number of] weeks. | ||
| + | |style="text-align:right;"|תכתו' לכל אחד לבדו כמה שהכתב מבדאי הריבי' לעי' ואח"כ המעות ואח"כ השובועו‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Take one of the sides, whichever you want, and multiply the amount of money by the [number of] weeks. | ||
| + | |style="text-align:right;"|ותשים צד אחד לפניך איזי שתרצה ותכפל המעות תוך השבועות | ||
| + | |- | ||
| + | | | ||
| + | ::Say: 9 times 1 is 9. | ||
| + | |style="text-align:right;"|ותאמ' ט'פ'א' שהוא ט‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Then, say: 9 times 4; you find 126. | ||
| + | ::<math>\scriptstyle{\color{blue}{9\times14=126}}</math> | ||
| + | |style="text-align:right;"|ואח"כ תאמ' טפ"ד ותמצא <s>הוא אז תחבר</s> וב"א | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color: blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | | ||7 | ||
| + | |- | ||
| + | | ||9 | ||
| + | |- | ||
| + | |1||4 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | | ||ז | ||
| + | |- | ||
| + | | ||ט | ||
| + | |- | ||
| + | |א||ד | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Do the same for the other side; you find 165. | ||
| + | ::<math>\scriptstyle{\color{blue}{11\times15=165}}</math> | ||
| + | |style="text-align:right;"|ותעשה ג"כ לצד השיני ותמצא הו"א | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color: blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |1||1 | ||
| + | |- | ||
| + | |1||5 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |א||א | ||
| + | |- | ||
| + | |א||ה | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Next, add 126 and 165 together; you find 291. Write it down, because this is the denominator. | ||
| + | ::<math>\scriptstyle{\color{blue}{126+165=291}}</math> | ||
| + | |style="text-align:right;"|אז תחבר אותם ליחד וב"א והו"א ותמצא אט"ב וזה תרשום לפניך כי הוא המור‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Then, multiply each, 126 and 165, by the interest: | ||
| + | |style="text-align:right;"|ואח"כ תכפול כל אחד ואחד וב"א והו"א תוך הריבית | ||
| + | |- | ||
| + | | | ||
| + | ::When you multiply 126 by 7, you find 882. | ||
| + | |style="text-align:right;"|וכשתכפול וב"א תוך הז' אז תמצא בח"ח | ||
| + | |- | ||
| + | | | ||
| + | ::Divide 882 by the 291 mentioned above; you find 291 is 3 times in 882. The 3 are the zehuvim that he has in his share and the 9 you have left from the division are the 9 greats of which 291 are one zahuv. | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{126\sdot7}{291}=\frac{882}{291}=3+\frac{9}{291}}}</math> | ||
| + | |style="text-align:right;"|ותחליק אט"ב הנזכרים לעיל מן בח"ח ותמצ' אט"ב תוך בח"ח ג"פ והג' הוא הזהו' שיהיו לו בחלקו וגם ט' נשאר לך מן החילק הוא הוי ט' גדולי' שעולי' אט"ב על אט"ב על אט"ב זהוב אחד | ||
| + | |- | ||
| + | | | ||
| + | ::Do the same with the other side: multiply it by 7; you find 1155. | ||
| + | |style="text-align:right;"|ותעשה ג"כ לצד השני ותיכפול הוא תוך הז' ותמצא ה"הא"א | ||
| + | |- | ||
| + | | | ||
| + | ::Divide 1155 also by the 291; you find 291 is 3 times in 1155, which are the zehuvim that he has in his share and you have 282 left that is less than 291 that are the greats of which 291 are one zahuv. Hence, we find that his share is 3 zehuvim and 282 greats, of which 291 are one zahuv. | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{165\sdot7}{291}=\frac{1155}{291}=3+\frac{282}{291}}}</math> | ||
| + | |style="text-align:right;"|ותחליק ג"כ אט"ב מן ה"הא"א ותמצא אט"ב תוך ה"אא"א ג"פ שהוא הזהו' שיהיו לו בחלקו וגם נשאר לך בח"ב שלא יכולים להשיג לכלל אט"ב שהוא גדולים שעולים אט"ב על זהו' אחד ונמצא שיהיו לזה בחלקו ג' זהו' ובח"ב גדולי' שעולי' אט"ב על זהוב אחד סליק דוק | ||
| + | |- | ||
| + | | | ||
| + | *If you are asked: how much is this circle? When you are told [the length of] the versine and half the chord. | ||
| + | |style="text-align:right;"|ואם נשאל לך על זה העיגול כמה כי יאמר לך החץ וחצי היתור אותו | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | [[File:Ṣifra - Circle.png|thumb|150px|center]] | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | [[File:ציפרא - מעגל.png|thumb|150px|center]] | ||
| + | |- | ||
| + | | | ||
| + | ::Then, say: how much is half the chord? It is 4 cubits. | ||
| + | |style="text-align:right;"|אז תאמר כמה היתור חצי הוא הד' אמות | ||
| + | |- | ||
| + | | | ||
| + | ::Say: 4 times 4 is 16. | ||
| + | |style="text-align:right;"|אז תאמ' ד'פ'ד' והוא ו"א | ||
| + | |- | ||
| + | | | ||
| + | ::Say: how much is the length of the versine? it is one cubit. | ||
| + | |style="text-align:right;"|ותאמ' כמה אורך החץ והוא אמה | ||
| + | |- | ||
| + | | | ||
| + | ::Divide the 16 by the cubit and say: how many 1's are in 16? It is 16. | ||
| + | |style="text-align:right;"|אז תחלק האמ' מן הו"א ותאמר כמה א' בתוך ו"א והוא ו"א והוא ו"א פ' בתוכה | ||
| + | |- | ||
| + | |? | ||
| + | |style="text-align:right;"|אז תעשה אורך החץ שהוא א' על הו"א אז יהיה ז"א | ||
| + | |- | ||
| + | | | ||
| + | ::Take the 16 and this is the length of the circle. | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{4\sdot4}{1}=\frac{16}{1}=16}}</math> | ||
| + | |style="text-align:right;"|וקח הו"א אורכו העיגול | ||
| + | |- | ||
| + | | | ||
| + | ::Always do the same: multiply [half] the chord [by itself], then divide the product by the versine. | ||
| + | |style="text-align:right;"|וכן תעשה לכל פעם תכפול היתור תחלק החץ מן הנכפל ודוק | ||
| + | |- | ||
| + | | | ||
| + | *I will explain to you a question called the ladder: if you are asked about a ladder that is ten cubits high, if we move the ladder up 2 cubits, how many cubits will it move from the wall below? like this: | ||
| + | |style="text-align:right;"|הנה אפרש לך שאלה שנקר' הסולם אם נשאל לך על סולם אחד שהיא גבוה עשרה אמות ואם נישג הסולם למעלה ב' אמות<br> | ||
| + | כמה אמות השיג למטה מחומה<br> | ||
| + | כמה זה | ||
| + | |- | ||
| + | | | ||
| + | ::Do as follow: | ||
| + | |style="text-align:right;"|אז תעשה כך | ||
| + | |- | ||
| + | | | ||
| + | ::Say: how much is the length of the ladder? Its length is ten [cubits]. | ||
| + | |style="text-align:right;"|תאמ' כמה אורך הסול' אורכה עשרה | ||
| + | |- | ||
| + | | | ||
| + | ::Say: 10 times 10 is 100. | ||
| + | |style="text-align:right;"|ותאמ' י'פ'י' שהי' ק‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Say: how long is the wall from the ground to the top of the ladder? It is 8 cubits. | ||
| + | |style="text-align:right;"|ותאמ' כמה אורך החומה מן הארץ עד ראש הסולם והיא ח' אמות | ||
| + | |- | ||
| + | | | ||
| + | ::Say: 8 times 8 is 64. | ||
| + | |style="text-align:right;"|ותאמ' ח'פ'ח' והוא ד"ו | ||
| + | |- | ||
| + | | | ||
| + | ::Subtract [64] from 100; 36 remains. | ||
| + | |style="text-align:right;"|ותחלק הח' מן <s>00</s> ה0'0'א' ונשאר ו"ג | ||
| + | |- | ||
| + | | | ||
| + | ::[Extract the root] of 36; the root of 36 is 6 and this is the length from the wall to the ladder on the ground. | ||
| + | ::<math>\scriptstyle{\color{blue}{\sqrt{10^2-8^2}=\sqrt{100-64}=\sqrt{36}=6}}</math> | ||
| + | |style="text-align:right;"|ותעשה שורת בהו'ג' והשורת בהוג הוא ו' זה אורך הקרקע מן החומה עד הסולם זליק | ||
| + | |- | ||
| + | | | ||
| + | *[Margin:] Here it is proven that the diagonal of the square of a cubit by a cubit is a cubit and two-fifths. | ||
| + | |style="text-align:right;"|בזה הוכיח דלאכח מה שבריבוע אמה על אמה שהאלכסון שלו אמ' ותרי חומשין | ||
| + | |- | ||
| + | | | ||
| + | :Because this is 10 cubits by 10 cubits: | ||
| + | |style="text-align:right;"|כי זה 0"א אמות על 0"א אמו' ודוק | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | [[File:Ṣifra - Square.png|thumb|200px|center]] | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | [[File:ציפרא - ריבוע.png|thumb|200px|center]] | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== <span style=color:Green>Moscow, Russian State Library, Ms. Guenzburg 714/4</span> ==== | ||
| + | |||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | *Question: here are many bulls one after another, meaning that every time I bought the first for a certain amount, I then bought the bull that follows it for double the first and likewise the third bull I bought also for double [the price] that I bought the one that preceded it and so the fourth bull and the fifth bull. | ||
| + | |style="text-align:right;"|‫<ref>82r</ref><big>שאלה</big> הנה שוורים הרבה בזה אחר זה ובענין זה בכל פעם כשקניתי הראשון בעד סך א' אז קניתי אותו שור של אחריו בעד כפל הראשון וכן השור השלישי קניתי ג"כ בעד כפל שקניתי אותו שלפניו וכן השור הד' וכן השור הה‫' | ||
| + | |- | ||
| + | | | ||
| + | :*For example: I bought the first for 1 zahuv, the second for 2 zehuvim, the third for 4 and so on. The question is how much is the total amount of these coins? | ||
| + | |style="text-align:right;"|כגון הא' קניתיו בעד א' זהוב הב' בב' זהובי' הג' בד' וכן כולם והנה השאלה כמה כל המעות הללו בסך אחת | ||
| + | |- | ||
| + | | | ||
| + | ::The answer to this example and others, in order to find, calculate and understand well, do it in this matter and in this way: | ||
| + | |style="text-align:right;"|<big>תשובה</big> זאת ועוד אחרת דוגמא זה למצוא ולחשוב ולעמוד על בוריו תעשה בענין זה ואופן זה | ||
| + | |- | ||
| + | | | ||
| + | ::First, look and understand how much is the first amount, for which you bought the first bull. | ||
| + | |style="text-align:right;"|בתחלה תראה ותבין כמה הוא סך הראשון שקנית בו השור הראשון | ||
| + | |- | ||
| + | | | ||
| + | ::Then, see also how much is twice the last amount, according to the multiplication chapter. | ||
| + | |style="text-align:right;"|ואח"כ תראה ג"כ כמה הוא הסך האחרון ב' פעמים בשער הכפל גדול | ||
| + | |- | ||
| + | | | ||
| + | ::Subtract from it the first amount, for which you bought the first bull, according to the subtraction chapter; and what you have left, when you subtract the first amount from it, is the total amount of coins, for which you bought all the bulls. | ||
| + | |style="text-align:right;"|ותחסר ממנו בשער החיסור הסך הראשון שקנית בו השור הראשון ומה שישאר בידך כשחסרת ממנו הסך הא' הוא סכום כל המעות שקנית בהם כל השוורים | ||
| + | |- | ||
| + | | | ||
| + | ::For the first example, when you bought the first for 1 zahuv, the second for 2 zehuvim, the third for 4, the fourth for 8, the fifth for 16, the sixth for 32, and the seventh for 64: | ||
| + | |style="text-align:right;"|והנה לך הדמיון כגון הראשון קנית בעד א' זהוב והשני בעד ב' והשלישי בעד ד' והרביעי בעד ח' והה' בעד י"ו והו' בעד ל"ב והז' בעד ס"ד | ||
| + | |- | ||
| + | | | ||
| + | ::You see that the last amount, for which you bought the seventh bull, i.e. the last bull, is 64 zehuvim. So, multiply according to the multiplication chapter: 2 times 64; it is 128. | ||
| + | |style="text-align:right;"|והנה תראה אתה שהסך האחרון שקנית בו השור הז' היינו השור האחרון הוא ס"ד זהובים א"כ תחשוב בשער הכפול הגדול ב' פעמים ס"ד ויהיה ‫<ref>82v</ref>ס"ד ויהיה קכ"ח | ||
| + | |- | ||
| + | | | ||
| + | ::Then, subtract from 128 the first amount, for which you bought the first bull, which is 1 zahuv; you have 128 left and this is the total amount for which you bought all the bulls. | ||
| + | |style="text-align:right;"|אז תחסר מן קכ"ח הסך הראשון שקנית בו השור הא' שהוא א' זהוב וישאר בידך קכ"ז זה כל כך מעות קנית כל השוורים | ||
| + | |- | ||
| + | |colspan="2" | | ||
| + | ::<math>\scriptstyle{\color{blue}{1+2+4+8+16+32+64=\left(2\sdot64\right)-1=128-1=127}}</math> | ||
| + | |- | ||
| + | | | ||
| + | ::Each time subtract the first number from the last number, after doubling it, not necessarily if the first number is 1: the same is true if the first number is 2, or 3, or 4, or whatever it is at the beginning; subtract it from the last number after you doubling it. Do not change this procedure, because it is the same and it is correct. | ||
| + | |style="text-align:right;"|וכן כל פעם תחסר מן הסך האחרון סך הראשון אחרי אשר כפלת אותו ב' פעמים ולאו דוקא אם הסך הראשון א' שהוא הדין אם הסך הא' ב' או ג' או ד' או כמה שהוא בתחלה תחסר מן הסך האחרון כשכפלת אותו ב"פ ואל תשנה מעניין זה כי חשבון א' הוא ואמת | ||
| + | |- | ||
| + | |colspan="2" | | ||
| + | ::<math>\scriptstyle{\color{OliveGreen}{q=2\longrightarrow S_n=2a_n-a_1}}</math> | ||
| + | |- | ||
| + | | | ||
| + | *Question: if you want to know any number you want in such a way that each time the next number is 4 times the previous number, as: 1, 4, 16, 256 and you want to know how much the sum is. | ||
| + | |style="text-align:right;"|<big>שאלה</big> אם תרצה לידע ולחשוב חשבון מה שתרצה בעניין זה בכל פעם אותו חשבון שלאחריו הוא ד' פעמים כמו אותו חשבון שלפניו כגון א' ד' י"ו ס"ד ס"ד רנ"ו ותרצה לידע ולחשוב כמה הוא סך אחד | ||
| + | |- | ||
| + | | | ||
| + | ::I will teach you the way so that you will succeed and become educated. | ||
| + | |style="text-align:right;"|אשכילך בדרך זו תלך למען תצליח ותשכיל | ||
| + | |- | ||
| + | | | ||
| + | ::I will show you the way: | ||
| + | |style="text-align:right;"|והנה אראך הדרך אשר תלך בה | ||
| + | |- | ||
| + | | | ||
| + | ::First, see what are the first number and the last number. | ||
| + | |style="text-align:right;"|בתחלה תראה ותבין ג"כ על הסך הראשון ועל הסך האחרון כמה הם | ||
| + | |- | ||
| + | | | ||
| + | ::You do not have to multiply the last number, as I showed you above, but take it as you find it and subtract the first number from it. | ||
| + | |style="text-align:right;"|אמנם אינו צריך לכפול סך האחרון כמו שהראיתיך לעיל . רק קח בידך כמו שתמצא אותו עתה ותחסר ממנו סך הראשון מן סך האחרון | ||
| + | |- | ||
| + | | | ||
| + | ::Then, you can divide what you have left into three equal parts and this is easy to understand for any educated person who has exposed his soul to this precious craft. | ||
| + | |style="text-align:right;"|אז תוכל לחלק הנשאר בידך לג' חלקים שוים וזה קל להבין לכל בר משכיל אשר הערה את נפשו למלאכה היקרה הזאת | ||
| + | |- | ||
| + | | | ||
| + | ::I will give you a short example and teach you through it, so that you can understand it and see with your own eyes that you can divide what you have left into three parts after you subtract the first number from it. | ||
| + | |style="text-align:right;"|כי אמשול לך ‫<ref>83r</ref>משל בדמיון קטן ואשכילך בו למען תבין ממנו ותראה בעיניך שתוכל לחלק הנשאר בידך לג' חלקים אחרי שחסרת ממנו הסך הראשון | ||
| + | |- | ||
| + | | | ||
| + | ::Here you have an example: as if you wish to know and calculate the sum of 1, 4, 16, 64, 256. | ||
| + | |style="text-align:right;"|והנה לך הדמיון כגון אם תרצה לידע ולחשוב בסך א' א' ד' י"ו ס"ד רנ"ו ‫[...] | ||
| + | |- | ||
| + | | | ||
| + | ::Now, you see that the last number is 256 and the first number is 1. You want to calculate the sum of all the numbers. Take the last number, which is 256, and subtract from it the last number, which is 1. You have 255 left. | ||
| + | |style="text-align:right;"|תראה עתה שהסך האחרון הוא רנ"ו והסך הא' הוא א' ותרצה לחשוב כל הסכומין בסך אחד אז קח הסך האחרון היינו רנ"ו ותחסר ממנו הסך הראשון היינו א' וישאר לך רנ"ה | ||
| + | |- | ||
| + | | | ||
| + | ::Then, divide according to the chapter of division: how many threes are there in 255? You find that the number is divided into three equal parts and you have no remainder after subtracting the first number from it, which is 1. If you do this, you find that there are 85 times 3 is 255, i.e. it is the third of 255, because 85 times 3 is 255. | ||
| + | |style="text-align:right;"|ואח"כ תחלק בשער החלוק כמה יש ברנ"ה הג' כי תמצא שיצא החשבון לג' חלקים שוים ולא ישאר לך מהם כלום אחר אשר חסרת ממנו הסך הראשון היינו א' ואם אתה עושה כן תמצא שיש בו פ"ה פעמים ג' ברנ"ה והיינו השליש מן הרנ"ה כי פ"ה פעמים ג' היינו רנ"ה | ||
| + | |- | ||
| + | | | ||
| + | ::Hence, you see that the number is divided into three equal parts after subtracting the first number from it. | ||
| + | |style="text-align:right;"|אם כן תראה עתה שיצא החשבון בג' חלקים שוים אחרי שחסרת ממנו הסך הראשון | ||
| + | |- | ||
| + | | | ||
| + | ::Then, take the third of 225, i.e. 85, and add it to 256, because this is the original last number, before you subtracted the first number from it. After adding, you find the result is [341] and this is the whole sum you wanted to know. When you sum up 1, 4, 16, 64, 256 you receive [341]. | ||
| + | |style="text-align:right;"|אח"כ קח אותו השליש מן רנ"ה דהיינו פ"ה וחבר אותו אל רנ"ו כי כן היה הסך האחרון מתחלה קודם שחסרת ממנו הסך הראשון ותמצא אחר החיבור שיצא לך תר"ל (?) והיינו כל החשבון אשר חפצת לידע כי כשתחבר ביחד א' ד' י"ו ס"ד ס"ד רנ"ו יעלה לך תר"ל (?) | ||
| + | |- | ||
| + | |colspan="2" | | ||
| + | ::<math>\scriptstyle{\color{blue}{1+4+16+64+256=\frac{256-1}{3}+256=\frac{255}{3}+256=85+256={\color{red}{341}}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | ::Let your your self understand and not forget to subtract the first number each time from the last number, so the result of the subtraction is divided into three equal parts. Then, you can take the part of 3, i.e. the third and add it to the original last number. | ||
| + | |style="text-align:right;"|ותן לבך להבין ולא לשכוח בכל ‫<ref>83v</ref>פעם לחסר החשבון הראשון מן סך האחרון ויצא אחר החיסור לג' חלקים שוים אח"כ תוכל ליקח החלק הג' היינו השליש ולחברו אל הסך האחרון כמו שהיה בתחלה | ||
| + | |- | ||
| + | | | ||
| + | ::Not necessarily if the first number is 1: the same is true if the first number is 2, or 3, or 4, or 5, or as much as you like. You can subtract it from the last number each time and you will receive each time after the subtraction the result is divided into three equal parts. Add this third to the last number as it was at the beginning and this is the sum. | ||
| + | |style="text-align:right;"|ולאו דוקא אם הסך הראשון א' הוא הדין אם הסך הראשון ב' או ג' או ד' או ה' או כמה שתרצה תוכל בכל פעם לחסר אותו מן הסך האחרון ויצא לך הסך בכל פעם אחר החיסור לג' חלקים שוים וחבור אותו השליש לסך האחרון כמו שהיה בתחלה וזהו חשבון ודוק תם | ||
| + | |- | ||
| + | |colspan="2" | | ||
| + | ::<math>\scriptstyle{\color{OliveGreen}{q=4\longrightarrow S_n=\frac{a_n-a_1}{3}+a_n}}</math> | ||
| + | |- | ||
| + | !<span style=color:Green>Give and Take Problems</span> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:market|627|9GcW}}A man went to the market; he doubled his money and spent 12 pešuṭim. | ||
| + | :He went to another market; he doubled what he had left and spent 12 pešuṭim. | ||
| + | :He went to yet another market; he doubled what he had left and spent 12 pešuṭim [...] He has nothing left. | ||
| + | :How much was the original amount of money he went with to the first market? | ||
| + | |style="text-align:right;"|‫<ref>84r</ref>אדם הלך לשוק וכפל מעותיו והוציא י"ב פשוטים<br> | ||
| + | ועוד הלך לשוק אחר וכפל הנשאר בידו והוציא י"ב פשוטים<br> | ||
| + | ועוד הלך לשוק אחר וכפל הנשאר בידו והוציא [י"ב פשוטים] [...] בידו כלום<br> | ||
| + | כמה היה הקרן שהוליך כמו בשוק הראשון{{#annotend:9GcW}} | ||
| + | |- | ||
| + | | | ||
| + | ::Do as follows: | ||
| + | |style="text-align:right;"|עשה כך | ||
| + | |- | ||
| + | | | ||
| + | ::For the first we should take a half from the 12 that he spent. | ||
| + | |style="text-align:right;"|בעבור הראשון נצטרך להוציא החצי מן הי"ב שהוציא | ||
| + | |- | ||
| + | | | ||
| + | ::For the second we take a quarter. | ||
| + | |style="text-align:right;"|ובעבור השני נקח הרביעי | ||
| + | |- | ||
| + | | | ||
| + | ::For the third we take an eighth, which is one pašuṭ and a half. | ||
| + | |style="text-align:right;"|ובעבור השלישי נקח השמינית מי"ב שהוא פשוט וחצי | ||
| + | |- | ||
| + | | | ||
| + | ::Subtract 1 pašuṭ a a half from 12; 10 and a half remain and this was original amount of money he went with to the first market. | ||
| + | ::<math>\scriptstyle{\color{blue}{12-\left(\frac{1}{8}\sdot12\right)=12-\left(1+\frac{1}{2}\right)=10+\frac{1}{2}}}</math> | ||
| + | |style="text-align:right;"|הסר א' פשוט וחצי מי"ב ישארו י' וחצי וכן היה הקרן שהוליך לשוק הראשון | ||
| + | |- | ||
| + | | | ||
| + | :According to this way you can do even if he goes to several markets: | ||
| + | |style="text-align:right;"|ועל זה הדרך תוכל לעשות ואם ילך לכמה שווקים | ||
| + | |- | ||
| + | | | ||
| + | ::For the fourth market we subtract one part of 16 from the 12 that he spent each time. | ||
| + | |style="text-align:right;"|כי בעבור השוק הרביעי נסיר מן הי"ב פ' שהיה מוציא בכל פעם חלק א' מי"ו | ||
| + | |- | ||
| + | | | ||
| + | ::For the fifth we subtract one part of 32. | ||
| + | |style="text-align:right;"|ובעבור החמישי נסיר חלק א' מל"ב | ||
| + | |- | ||
| + | | | ||
| + | ::For the sixth we subtract one part of 64. | ||
| + | |style="text-align:right;"|ובעבור השישי נסיר חלק א' מס"ד | ||
| + | |- | ||
| + | | | ||
| + | :As many markets you have, so you double what you have, then divide what he spent by the double and subtract the quotient from what he spent; and this is the required. | ||
| + | |style="text-align:right;"|וכאשר תרבה השווקים כן תכפול מה שיש בידך ועל הכפול חלק מה שהוציא ומה שיעלה בחילוק הסר ממה שהוציא והוא המבוקש | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:gatekeeper|651|lOQx}}A man went to the orchard to pick apples and he has to pick from them so that he will give the inner keeper of the orchard half the apples he collected and one more, to the second gatekeeper of the second gate he has to give half of the apples left in his hand and one more and so on he has [to give to all the gatekeepers] of the six gates of the orchard, that is, to give each gatekeeper a half and one more [...] apples no less and no more. | ||
| + | |style="text-align:right;"|<big>אדם</big> א' הלך לפרדס א' ללקוט תפוחים ויש לו ללקוט מהם כל כך שיתן לשוער הפנימי ‫<ref>84v</ref>של הפרדס חצי התפוחים שלקט וא' יותר ולשוער השני מן השער הב' יש לו ליתן החצי מן התפוחים שנשארו בידו וא' יותר וכן יש לו [ליתן] [...] לששה שערים שיש לפרדס דהיינו לתת לכל שוער החצי וא' יותר [...] תפוחים לא פחות ולא יותר עשה כך{{#annotend:lOQx}} | ||
| + | |- | ||
| + | | | ||
| + | ::Take number 5 that he must bring and add 1 to it; it is 6. | ||
| + | |style="text-align:right;"|תפוש מספר הה' שהוא חייב להביא ותוסיף עליו א' הרי ו‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Double 6; it is 12 for one gate. | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(5+1\right)\sdot2=6\sdot2=12}}</math> | ||
| + | |style="text-align:right;"|כפול ו' ויהא י"ב הרי שער א‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Add 1 to it; it is 13. | ||
| + | |style="text-align:right;"|הוסיף עליו א' הרי י"ג | ||
| + | |- | ||
| + | | | ||
| + | ::Double it; it is 26 for two gates. | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(12+1\right)\sdot2=13\sdot2=26}}</math> | ||
| + | |style="text-align:right;"|כפול אותם ויהיו כ"ו הרי ב' שערים | ||
| + | |- | ||
| + | | | ||
| + | ::Add 1 to it; it is 27. | ||
| + | |style="text-align:right;"|תוסיף עליו א' ויהיו כ"ז | ||
| + | |- | ||
| + | | | ||
| + | ::Double it; it is 54 for three gates. | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(26+1\right)\sdot2=27\sdot2=54}}</math> | ||
| + | |style="text-align:right;"|כפול אותם ויהיו נ"ד הרי ג' שערים | ||
| + | |- | ||
| + | | | ||
| + | ::Add 1 to it; it is 55. | ||
| + | |style="text-align:right;"|תוסיף עליהם א' ויהיו נ"ה | ||
| + | |- | ||
| + | | | ||
| + | ::Double it; it is 110 for four gates. | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(54+1\right)\sdot2=55\sdot2=110}}</math> | ||
| + | |style="text-align:right;"|כפול אותם ויהיו ק"י הרי ד' שערים | ||
| + | |- | ||
| + | | | ||
| + | ::Add 1 to it; it is 111. | ||
| + | |style="text-align:right;"|תוסיף עליהם א' ויהיו קי"א | ||
| + | |- | ||
| + | | | ||
| + | ::Double it; it is 222 for five gates. | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(110+1\right)\sdot2=111\sdot2=222}}</math> | ||
| + | |style="text-align:right;"|כפול אותם ויהיו רכ"ב הרי ה' שערים | ||
| + | |- | ||
| + | | | ||
| + | ::Add 1 to it; it is 223. | ||
| + | |style="text-align:right;"|תוסיף עליהם א' ויהיו רכ"ג | ||
| + | |- | ||
| + | | | ||
| + | ::Double it; it is 446 for six gates. | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(222+1\right)\sdot2=223\sdot2=446}}</math> | ||
| + | |style="text-align:right;"|כפול אותם ויהיו תמ"ו הרי ו' שערים | ||
| + | |- | ||
| + | | | ||
| + | ::So, he has to collect a total of 446 apples and 5 apples are left for him to bring no less and no more. | ||
| + | |style="text-align:right;"|הרי שיש לו ללקט תמ"ו תפוחים בין הכל וישארו בידו ה' תפוחים להביא לא פיחות ולא יותר | ||
| + | |- | ||
| + | | | ||
| + | *If he has pick from them so that he will give the inner keeper half of what he has collected plus one more, to the second gatekeeper half of what is left plus 2 more, to the third gatekeeper half of what is left plus 3 more, to the fourth gatekeeper half of what is left plus 4 more, and he has to bring 4 apples no less and no more. | ||
| + | |style="text-align:right;"|ואם יש לו ללקט מהם כל כך שיתן לשוער הפנימי החצי ממה שלקט וא' יותר ולשוער הב' חצי הנשאר וב' יותר ולשוער הג' חצי הנשאר וג' יותר ‫<ref>85r</ref>ולשוער הד' חצי הנשאר וד' יותר וד' תפוחים יש לו להביא לא פחות ולא יותר | ||
| + | |- | ||
| + | | | ||
| + | ::Do as follows: | ||
| + | |style="text-align:right;"|עשה כך | ||
| + | |- | ||
| + | | | ||
| + | ::Take the number 4 that he has to bring, add 4 to it that he has to give the gatekeeper [...] | ||
| + | ::<math>\scriptstyle{\color{OliveGreen}{\left(4+4\right)\sdot2=8\sdot2=16}}</math> | ||
| + | |style="text-align:right;"|תפוס מספר הד' שיש לו להביא הוסף עליהם ד' שיש לו להוסיף לשו[ער] [...] שער א‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Add 3 to it; it is 19. | ||
| + | |style="text-align:right;"|הוסף [עליהם ג' וי]היו י"ט | ||
| + | |- | ||
| + | | | ||
| + | ::Double it; it is 38 for two gates. | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(16+3\right)\sdot2=19\sdot2=38}}</math> | ||
| + | |style="text-align:right;"|כפלם ויהיו ל"ח הרי ב' שערים | ||
| + | |- | ||
| + | | | ||
| + | ::Add 2 to it; it is 40. | ||
| + | |style="text-align:right;"|הוסף עליהם ב' ויהיו מ‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Double it; it is 80 for three gates. | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(38+2\right)\sdot2=40\sdot2=80}}</math> | ||
| + | |style="text-align:right;"|כפלם ויהיו פ' הרי ג' שערים | ||
| + | |- | ||
| + | | | ||
| + | ::Add 1 to it; it is 81. | ||
| + | |style="text-align:right;"|הוסף עליהם א' יהיו פ"א | ||
| + | |- | ||
| + | | | ||
| + | ::Double it; it is 162 for four gates. | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(80+1\right)\sdot2=81\sdot2=162}}</math> | ||
| + | |style="text-align:right;"|כפלם ויהיו קס"ב הרי ד' שערים | ||
| + | |- | ||
| + | | | ||
| + | ::So, he collected 162 apples and 4 apples were left for him. | ||
| + | |style="text-align:right;"|והתפוחים שלקט היו קס"ב והנשארים בידו ד' תפוחים | ||
| + | |- | ||
| + | !<span style=color:Green>Find a Quantity Problems - Whole from Parts</span> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:lance|650|7Bg8}}A lance is embedded one-third and one-quarter in the ground, and it is 80 cubits above the ground. | ||
| + | :How many cubits are the whole lance? | ||
| + | :<math>\scriptstyle\frac{1}{3}X+\frac{1}{4}X+80=X</math> | ||
| + | |style="text-align:right;"|<big>חנית</big> מעוכה בארץ שלישיתה ורביעיתה ולמעלה מן הארץ היא פ' אמה<br> | ||
| + | כמה אמות היא בין כולה{{#annotend:7Bg8}} | ||
| + | |- | ||
| + | | | ||
| + | :Do as follows: | ||
| + | |style="text-align:right;"|עשה כך | ||
| + | |- | ||
| + | | | ||
| + | ::Find a number that has a third and a quarter; it is 12. | ||
| + | |style="text-align:right;"|תמצא מספר שיש לו שליש ורביע והוא י"ב | ||
| + | |- | ||
| + | | | ||
| + | ::A third and aquarter of 12 are 7 and it is the [part] that is embedded in the ground. | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(\frac{1}{3}\sdot12\right)+\left(\frac{1}{4}\sdot12\right)=7}}</math> | ||
| + | |style="text-align:right;"|ושליש ורביע מי"ב הוא ז' והוא התחוב בארץ | ||
| + | |- | ||
| + | | | ||
| + | ::5 remains above the ground. | ||
| + | |style="text-align:right;"|ולמעלה מן הארץ נשאר ה‫' | ||
| + | |- | ||
| + | | | ||
| + | ::So, say: if 5 equals 12, how much is 20 equal? | ||
| + | |style="text-align:right;"|אם כך אמור כך אם ה' שווה י"ב כמה ישוה כ‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Multiply 20 times 12; the result is 240. | ||
| + | |style="text-align:right;"|כפול אותם כ"פ י"ב יעלו ר"מ | ||
| + | |- | ||
| + | | | ||
| + | ::Divide it by 5; the result of division is 48. | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{20\sdot12}{5}=\frac{240}{5}=48}}</math> | ||
| + | |style="text-align:right;"|חלקם על ה' יעלו מ"ח בחלוק | ||
| + | |- | ||
| + | | | ||
| + | :Hence, the length of the lance is 48 cubits. | ||
| + | |style="text-align:right;"|א"כ אורך החנית מ"ח אמות | ||
| + | |- | ||
| + | | | ||
| + | *Reuven hired Shimon to build him a house in 30 days. | ||
| + | :For a day working in construction he will earn 9 liṭra, and for a day he does not work, he will lose 4 liṭra. | ||
| + | :At the end of 30 days he did not earn or lose. | ||
| + | :How many days did he work and how many days he did not work? | ||
| + | :<math>\scriptstyle3x=\left(30-x\right)\sdot4</math> | ||
| + | |style="text-align:right;"|<big>ראובן</big> שכר שמעון שיבנה לו בית א' בל' יום<br> | ||
| + | והיום שיעבוד בבנין ירויח ג' ליט' והיום שלא יעבוד יפסיד ד' ליט‫'<br> | ||
| + | ובסוף ל' יום לא הרויח ולא הפסיד כלום<br> | ||
| + | כמה ימים עבד וכמה ימים לא ‫<ref>85v</ref>עבד | ||
| + | |- | ||
| + | | | ||
| + | ::Do as follows: there is no doubt that what he earns in 4 days at 3 liṭra a day, he loses in 3 days at 4 liṭra a day. | ||
| + | |style="text-align:right;"|עשה כך אין ספק כי מה שירויח בד' ימים לחשבון ג' ליט' ליום יפסיד בג' ימים לחשבון ד' ליט' ליום | ||
| + | |- | ||
| + | | | ||
| + | ::Add 4 to 3; it is 7. | ||
| + | |style="text-align:right;"|חבר ד' עם הג' יהיו ז‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Multiply 3 times 30; it is 90. | ||
| + | |style="text-align:right;"|כפול ג"פ ל' יהיו צ‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Divide it by 7; the result is 12 days and 6 parts of 7 and they are the days in which he did not work. | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{3\sdot30}{3+4}=\frac{90}{7}=12+\frac{6}{7}}}</math> | ||
| + | |style="text-align:right;"|חלקם על [ז' יעלו] ‫<ref>marg.</ref>[י"ב ימים וו' חלקים של ז' והם הימים שלא עבד בה | ||
| + | |- | ||
| + | | | ||
| + | ::Then, multiply 4 times 30; the result is 120. | ||
| + | |style="text-align:right;"|ואחר הכפיל ד"פ ל' ויעלו ק"כ | ||
| + | |- | ||
| + | | | ||
| + | ::Divide it by 7; the result is 17 days and 1 part of 7 of a day and they are the days in which he worked. | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{4\sdot30}{3+4}=\frac{120}{7}=17+\frac{1}{7}}}</math> | ||
| + | |style="text-align:right;"|חלקם על ז' ויעלו י"ז יום וחלק א' מז' ביום‫] וכן ימים עבד בה | ||
| + | |- | ||
| + | | | ||
| + | *If it is said that for a day working in building the house he will earn 36 pešuṭim, for a day he does not work he will lose 41 pešuṭim and at the end of 30 days he did not earn or lose. | ||
| + | :<math>\scriptstyle36x=\left(30-x\right)\sdot41</math> | ||
| + | |style="text-align:right;"|ואם יאמר כי היום שיעבוד בבית ירויח ל"ו פשוט והיום שלא יעבוד יפסיד מ"א פשוטים ובסוף ל' יום לא הרויח ולא הפסיד כלום | ||
| + | |- | ||
| + | | | ||
| + | ::Do as follows: there is no doubt that what he earns in 41 days at 36 pešuṭim a day, he loses in 36 days at 41 pešuṭim a day. | ||
| + | |style="text-align:right;"|עשה כך אין ספק כי מה שירויח במ"א יום לחשבון ל"ו פשוטים ליום יפסיד בל"ו יום לחשבון מ"א פשוטים ליום | ||
| + | |- | ||
| + | | | ||
| + | ::Add 36 to 41; the result is 77. | ||
| + | |style="text-align:right;"|תחבר ל"ו עם מ"א יעלו ע"ז | ||
| + | |- | ||
| + | | | ||
| + | ::Multiply 36 times 30; you receive one thousand and 80. | ||
| + | |style="text-align:right;"|כפול ל"ו פעמים ל' <s>יהיו לך י"ד יום שלמים</s> יעלה לך אלף ופ‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Divide it by 77; the result of division is 14 whole days and 2 parts of 77 of a day and these are the days he did not work in construction. | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{36\sdot30}{36+41}=\frac{1080}{77}=14+\frac{2}{77}}}</math> | ||
| + | |style="text-align:right;"|חלקם על ע"ז ויעלו בחלוק י"ד יום שלם וב' חלקים מע"ז ביום וכך ימים לא עבד בבית | ||
| + | |- | ||
| + | | | ||
| + | ::Multiply also 41 times 30; the result is one thousand and 230. | ||
| + | |style="text-align:right;"|ועוד כפול מ"א פעמים ל' ויעלה אלף ור"ל | ||
| + | |- | ||
| + | | | ||
| + | ::Divide it by 77; the result of division is 15 whole days and 75 parts of 77 of a day. | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{41\sdot30}{36+41}=\frac{1230}{77}=15+\frac{75}{77}}}</math> | ||
| + | |style="text-align:right;"|חלקם על ע"ז ויעלו בחלוק ט"ו ימים שלמים וע"ה חלקים מע"ז ביום | ||
| + | |- | ||
| + | !<span style=color:Green>Divide a Quantity - Sharing Food</span> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | *Two men sat down to eat. | ||
| + | :One had two loaves of bread and the second had three loaves of bread. | ||
| + | :A third came and ate with them. | ||
| + | :The three ate the five loaves of bread and after they ate, the third, who ate with them, gave the other two five pešiṭim. | ||
| + | :[How should they share the five pešiṭim]? | ||
| + | :<math>\scriptstyle\left[\left(2-\frac{5}{3}\right)\sdot X\right]+\left[\left(3-\frac{5}{3}\right)\sdot X\right]=5</math> | ||
| + | |style="text-align:right;"|‫<ref>86r</ref><big>ב'</big> אנשים היו יושבין לאכול<br> | ||
| + | לאחד היו ב' לחמים ולשני ג' לחמים<br> | ||
| + | בא אדם שלישי ואכל עמהם<br> | ||
| + | ואכלו בין שלשתם אלו הה' לחמים<br> | ||
| + | לאחר שאכלו נתן אותו השלישי שאכל עמהם לאותם השנים ה' פשוטים [שיחלקום] ביניהם ‫[...] | ||
| + | |- | ||
| + | | | ||
| + | ::How much of the whole bread did each of them eat? | ||
| + | |style="text-align:right;"|כמה חלק מכל לחם אכל כל א' מהם | ||
| + | |- | ||
| + | | | ||
| + | ::One loaf of bread and 2-thirds of a loaf of bread. | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{5}{3}=1+\frac{2}{3}}}</math> | ||
| + | |style="text-align:right;"|א' לחם וב' שלישיות מלחם | ||
| + | |- | ||
| + | | | ||
| + | ::So, the one who had two loaves of bread and ate one loaf of bread and 2-thirds lost only one-third of a loaf of bread. | ||
| + | ::<math>\scriptstyle{\color{blue}{2-\left(1+\frac{2}{3}\right)=\frac{1}{3}}}</math> | ||
| + | |style="text-align:right;"|א"כ אותו שהיו לו ב' לחמים ואכל א' לחם וב' שלישיות לא הפסיד כי אם שליש לחם | ||
| + | |- | ||
| + | | | ||
| + | ::The one who had three loaves of bread and ate one loaf of bread and 2-thirds lost one loaf of bread and one-third that are 4-thirds of a loaf of bread. | ||
| + | ::<math>\scriptstyle{\color{blue}{3-\left(1+\frac{2}{3}\right)=1+\frac{1}{3}=\frac{4}{3}}}</math> | ||
| + | |style="text-align:right;"|ואותו שהיו לו ג' לחמים ואכל א' לחם וב' שלישיות הפסיד א' לחם ושליש שהם ד' שלישיות לחם | ||
| + | |- | ||
| + | | | ||
| + | ::Therefore, the one who had two loaves of bread will take 1 pašuṭ, because he lost a third of a loaf of bread. | ||
| + | |style="text-align:right;"|א"כ אותו שהיו לו ב' לחמים יקח א' פשוט כי הפסיד שליש לחם | ||
| + | |- | ||
| + | | | ||
| + | ::The one who had three loaves of bread will take 4 pešuṭim, because he lost one loaf of bread and a third that are 4-thirds of a loaf of bread. | ||
| + | |style="text-align:right;"|ואותו שהיו לו ג' לחמים יקח ד' פשוטים כי הפסיד לחם א' ושליש שהם ד' שלישי לחם | ||
| + | |- | ||
| + | !<span style=color:Green>Motion Problem - To and From - an Ant Climbing a Tower</span> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | *A tower is 20 cubits tall. An ant wants to climb up. Every day it climbs up one third of a cubit and every night it goes down a quarter of a cubit. How much further up it moves each day and in how many days it will reach to the top? | ||
| + | :<math>\scriptstyle\frac{1}{3}X-\frac{1}{4}X=20</math> | ||
| + | |style="text-align:right;"|<big>מגדל</big> שהוא גבוה כ' אמה ונמלה א' רוצה לעלות למעלה ובכל יום עולה שליש אמה ובכל לילה יורדה רביע אמה כמה יתרון יש לה בכל יום ובכמה ימים תעלה למעלה | ||
| + | |- | ||
| + | | | ||
| + | ::First, say: by how much is the third greater than the quarter? By 1 part of 12. | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{1}{3}-\frac{1}{4}=\frac{1}{12}}}</math> | ||
| + | |style="text-align:right;"|אמור תחלה כמה הוא יותר השליש מן הרביע א' חלק מי"ב | ||
| + | |- | ||
| + | | | ||
| + | ::So, in 12 days it climbs 1 cubit. | ||
| + | |style="text-align:right;"|הרי שבי"ב ימים היא עולה א' אמה | ||
| + | |- | ||
| + | | | ||
| + | ::Since the tower is 20 cubits tall, say: 12 times 20 is 240. Hence, in 240 days it reaches to the top of the tower. | ||
| + | ::<math>\scriptstyle{\color{blue}{x=12\sdot20=240}}</math> | ||
| + | |style="text-align:right;"|ובעבור שהמגדל היא גבוה כ' אמות אמור י"ב פעמים כ' הרי ר"מ הרי שבר"מ ימים היא עולה לראש המגדל | ||
| + | |- | ||
| + | !<span style=color:Green>How Much Problem – Money</span> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | *You have some money. You take a third, a quarter, and a fifth of it and they are nine pešuṭim. How much remains? | ||
| + | :<math>\scriptstyle X-\left(\frac{1}{3}X+\frac{1}{4}X+\frac{1}{5}X\right)=9</math> | ||
| + | |style="text-align:right;"|<big>הרי</big> שיש לך מעות והוצאת מהם השליש והרביע ‫<ref>86v</ref>והחומש והם ט' פשוטים<br> | ||
| + | כמה יהיו הנשארים | ||
| + | |- | ||
| + | | | ||
| + | ::First, say: a third, a quarter and a fifth are found in 60. The third is 20; the quarter is 15 and the fifth is 12. Their sum is 47. | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(\frac{1}{3}\sdot60\right)+\left(\frac{1}{4}\sdot60\right)+\left(\frac{1}{5}\sdot60\right)=20+15+12=47}}</math> | ||
| + | |style="text-align:right;"|אמור תחלה שליש ורביע וחומש ימצאו בס' השליש הוא כ' הרביע הוא ט"ו החומש הוא י"ב וכללם יהיו מ"ז | ||
| + | |- | ||
| + | | | ||
| + | ::So, the third, the quarter, and the fifth that are 9 pešuṭim are 47 parts of 60. | ||
| + | |style="text-align:right;"|הרי שהשליש והרביע והחומש שהם ט' פשוטים הם מ"ז חלקים מס‫' | ||
| + | |- | ||
| + | | | ||
| + | ::We find that the remainder is 13 parts of 60. | ||
| + | ::<math>\scriptstyle{\color{OliveGreen}{1-\frac{47}{60}=\frac{13}{60}}}</math> | ||
| + | |style="text-align:right;"|נמצא שהנשאר [...] י"ג חלקים מס‫' | ||
| + | |- | ||
| + | | | ||
| + | ::<span style=color:Green>'''Rule of Three'''</span>: So, say: if 47 parts of 60 are equal to 9 pešuṭim, how much are 13 parts of 60 equal to? | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{47}{60}:9=\frac{13}{60}:X}}</math> | ||
| + | |style="text-align:right;"|ולכן אמור אם מ"ז חלקים מס' שוים ט' פשוטים י"ג חלקים מס' כמה שוים | ||
| + | |- | ||
| + | | | ||
| + | ::Say: 13 times 9 is 117. | ||
| + | |style="text-align:right;"|אמור י"ג פעמים ט' הם קי"ז | ||
| + | |- | ||
| + | | | ||
| + | ::Divide it by 47; the result is [2] and 23 parts of 47. | ||
| + | ::<math>\scriptstyle{\color{blue}{X=\frac{13\sdot9}{47}=\frac{117}{47}=2+\frac{23}{47}}}</math> | ||
| + | |style="text-align:right;"|חלקם במ"ז יבואו כ"ג חלקים ממ"ז | ||
| + | |- | ||
| + | | | ||
| + | ::We find that there were 11 pešuṭim and 23 parts of 47 in the purse. He took out 9 and what remained was 2 and 23 parts of 47. | ||
| + | |style="text-align:right;"|נמצא שהיו בכיס י"א פשוטים וכ"ג חלקים ממ"ז ומה שהוציא הוא ט' ומה שנשאר היו ב' וכ"ג חלקים ממ"ז | ||
| + | |- | ||
| + | | | ||
| + | ::The same for any number you want. | ||
| + | |style="text-align:right;"|וכן לכל חשבון שתרצה | ||
| + | |- | ||
| + | | | ||
| + | *A man has a jug that holds 8 cups of wine and he wants to divide its content between two people, giving each 4 cups, but he has only two jugs – one that holds 3 cups and the other that holds 5 [cups]. | ||
| + | :How will he divide it, giving each 4 [cups]? | ||
| + | |style="text-align:right;"|<big>אדם</big> א' יש לו קנקן שמחזיק ח' כוסות של יין ורוצה לחלקו לשני אנשים וליתן לכל א' ד' כוסות ואין לו רק שני כלים שהא' מחזיק ג' כוסות וא' ה‫'<br> | ||
| + | היאך יעשה לחלק אותם וליתן ד' לכל א‫' | ||
| + | |- | ||
| + | | | ||
| + | ::First, he fills the [small] jug that holds 3 cups and pours [its content] into the [medium] jug that holds 5 cups. | ||
| + | |style="text-align:right;"|תחלה ימלא הכלי שמחזיק ג' כוסות וישים אותם בתוך הכלי המחזיק ה‫' | ||
| + | |- | ||
| + | | | ||
| + | ::<span style=color:green>3 cups - medium jug<br>5 cups - large jug</span> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | ::Then, he fills the [small] jug that holds 3 cups once more, and pours [its content] into the [medium] jug that holds 5 cups, so the [medium] jug that holds 5 cups is full and the [small] jug that holds 3 cups has one cup left. | ||
| + | |style="text-align:right;"|ואח"כ ימלא פעם אחר הכלי המחזיק ג' ויריק כמו כן באותו הכלי המחזיק ה' הרי שהכלי המחזיק ה' מלא ונשאר בכלי המחזיק ג' כוס א‫' | ||
| + | |- | ||
| + | | | ||
| + | ::<span style=color:green>1 cup - small jug<br>5 cups - medium jug<br>[2 cups - large jug]</span> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | ::Next, he pours [the content of the medium] jug that holds 5 cups into the [large] jug that holds 8 cups. We find that the large jug has 7 cups and the [small] jug that holds 3 cups has 1 cup. | ||
| + | |style="text-align:right;"|אח"כ יריק הכלי מלא של ה' כוסות בתוך הקנקן המחזיק ח' ונמצא ‫<ref>87r</ref>שבתוך הקנקן גדול יש בו ז' כוסות ובכלי המחזיק ג' יש בו כוס א‫' | ||
| + | |- | ||
| + | | | ||
| + | ::<span style=color:green>1 cup - small jug<br>7 cups - large jug</span> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | ::Then, he pours [the content of the small] jug that holds 3 cups, which has 1 cup, into the [medium] jug that holds 5 cups, refills the [small] jug that holds 3 cups from the [large] jug that holds 8 cups and pours [its content] into the [medium] jug that holds 5 cups. We now find that there are 4 cups in the [large] jug that holds 8 cups and 4 cups in the [medium] jug that holds 5 cups. So, the wine is divided evenly. | ||
| + | |style="text-align:right;"|אח"כ יריק הכלי של ג' כוסות שיש בו כוס א' בתוך הכלי המחזיק ה' ויחזור וימלא הכלי המחזיק ג' מתוך הכלי המחזיק ח' ויריק בתוך הכלי המחזיק ה' ונמצא עכשיו ד' כוסות בתוך הכלי המחזיק ח' וד' כוסות בתוך הכלי המחזיק ה' ועם זה היין חלוק בשוה | ||
| + | |- | ||
| + | | | ||
| + | ::<span style=color:green>4 cups - medium jug<br>4 cups - large jug</span> | ||
| + | | | ||
| + | |- | ||
| + | !<span style=color:Green>Joint Purchase Problem - If You Give Me - Amounts of Money</span> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | *Three friend, one said to his two friends: know that all I have in my purse with a half of what the both of you have is 60. The second answers and says: all I have in my purse and a quarter of what the both of you have is 60. The third answers: all I have in my purse and a third of what the both of you have is 60. | ||
| + | |style="text-align:right;"|<big>שלשה</big> חברים היו ואמר הא' לשני חבריו דעו כי כל מה שבכיסי והחצי משניכם עולה ס' ויען השני ויאמר כל מה שבכיסי ורביע משניכם עולה ס' ויען השלישי כל מה שבכיסי ושליש משניכם עולה ס‫' | ||
| + | |- | ||
| + | |colspan="2"| | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle X+\left[\frac{1}{2}\sdot\left(Y+Z\right)\right]=60\\\scriptstyle Y+\left[\frac{1}{4}\sdot\left(X+Z\right)\right]=60\\\scriptstyle Z+\left[\frac{1}{3}\sdot\left(X+Y\right)\right]=60\end{cases}</math> | ||
| + | |- | ||
| + | | | ||
| + | :*<span style=color:Green>'''False Position'''</span>: we found 3 numbers - 5, 11 and 13, which are a sort of [an answer to] this question. | ||
| + | |style="text-align:right;"|מצאנו ג' מספרים והם ה' י"א וי"ג שהם כעין השאלה הזאת | ||
| + | |- | ||
| + | |colspan="2"| | ||
| + | ::<math>\scriptstyle{\color{blue}{\begin{cases}\scriptstyle X_1=5\\\scriptstyle Y_1=11\\\scriptstyle Z_1=13\end{cases}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | ::For, 5 says to 11 and 13: me and a half of both of you is 17. | ||
| + | ::11 says to 5 and 13: me and a third of both of you is 17. | ||
| + | ::13 says to 5 and 11: me and a quarter of you is 17. | ||
| + | |style="text-align:right;"|כי ה' אומר לי"א ולי"ג אני וחצי שניכם י"ז<br> | ||
| + | וי"א אומר לה' ולי"ג אני ושליש שניכם י"ז<br> | ||
| + | וי"ג אומר לה' ולי"א אני ורביע שניכם י"ז | ||
| + | |- | ||
| + | |colspan="2"| | ||
| + | ::<math>\scriptstyle{\color{blue}{\begin{cases}\scriptstyle X_1+\left[\frac{1}{2}\sdot\left(Y_1+Z_1\right)\right]=17\\\scriptstyle Y_1+\left[\frac{1}{4}\sdot\left(X_1+Z_1\right)\right]=17\\\scriptstyle Z_1+\left[\frac{1}{3}\sdot\left(X_1+Y_1\right)\right]=17\end{cases}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | ::<span style=color:Green>'''Rule of Three'''</span>: you see that if they would have said: "the sum of all three of us is 17", the first would have 5, the second 11 and the third 13. | ||
| + | |style="text-align:right;"|והנך רואה שאלו אמרו מחובר שלשתנו י"ז יהיה לא' ה' ולשני י"א ולשלישי י"ג | ||
| + | |- | ||
| + | | | ||
| + | ::Now, that they say: "the sum of all three of us together is 60", we turn to the ratios and say: | ||
| + | |style="text-align:right;"|ועכשיו שאמרו {{#annot:term|388,1220|sXvR}}מחובר{{#annotend:sXvR}} שלשתנו יחד ס' נשוב לערכים ונאמר | ||
| + | |- | ||
| + | | | ||
| + | ::As the ratio of 5 to 17 so is the ratio of the smaller to 60, i.e. the one who asked a half of his friends. | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{5}{17}=\frac{X}{60}}}</math> | ||
| + | |style="text-align:right;"|כערך ה' לי"ז כן ערך הקטן אל ס' הוא ששאל לחבריו החצי | ||
| + | |- | ||
| + | | | ||
| + | ::As the ratio of 11 to 17 so is the ratio of the second to 60, i.e. the one who asked a third of his friends. | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{11}{17}=\frac{Y}{60}}}</math> | ||
| + | |style="text-align:right;"|וכערך י"א לי"ז כן ערך השני לס' והוא ששאל ‫<ref>87v</ref>לחבריו השליש | ||
| + | |- | ||
| + | | | ||
| + | ::As the ratio of 13 to 17 so is the ratio of the greater to 60, i.e. the one who asked a quarter of his friends. | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{13}{17}=\frac{Z}{60}}}</math> | ||
| + | |style="text-align:right;"|וכערך י"ג לי"ז כן ערך הגדול אל ס' והוא ששאל לחביריו הרביע | ||
| + | |- | ||
| + | | | ||
| + | ::Take the ratio for all the amounts stated by the three, set 5, 11, and 13 as the denominators and the procedure of the rule of three is as follows: | ||
| + | |style="text-align:right;"|וכן תערוך לכל הסכומים שיאמרו שלשתם תשים המורה ה' וי"א וי"ג ודרך הערכים הוא כענין | ||
| + | |- | ||
| + | | | ||
| + | ::Say: if, when the sum is 17, the share of the smaller is 5, how much is his share, when the sum is 60? | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{17}{5}=\frac{60}{X}}}</math> | ||
| + | |style="text-align:right;"|אמור אם בעת שהמחובר הוא י"ז יהיה חלק הקטן ה' בהיות המחובר ס' כמה הם חלקיו | ||
| + | |- | ||
| + | | | ||
| + | ::Say: 60 times 5 is 300. | ||
| + | |style="text-align:right;"|אמור ספ"ה הוא ש‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Divide it by 17; it is 17 and 11 parts of 17. | ||
| + | ::<math>\scriptstyle{\color{blue}{X=\frac{60\sdot5}{17}=\frac{300}{17}=17+\frac{11}{17}}}</math> | ||
| + | |style="text-align:right;"|וחלקם בי"ז יהיו י"ז וי"א חלקים מי"ז | ||
| + | |- | ||
| + | | | ||
| + | ::To know the share of the middle, say: if, when the sum is 17, the share of the middle is 11, how much is his share, when the sum is 60? | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{17}{11}=\frac{60}{Y}}}</math> | ||
| + | |style="text-align:right;"|ולדעת החלק האמצעי אמור אם בעת שהמחובר הוא י"ז שוה חלק האמצעי י"א בהיות המחובר ס' כמה הם חלקו | ||
| + | |- | ||
| + | | | ||
| + | ::Say: 60 times 11 is 660. | ||
| + | |style="text-align:right;"|אמור ס"פ י"א הם תר"ס | ||
| + | |- | ||
| + | | | ||
| + | ::Divide it by 17; it is 38 and 14 parts of 17. | ||
| + | ::<math>\scriptstyle{\color{blue}{Y=\frac{60\sdot11}{17}=\frac{660}{17}=38+\frac{14}{17}}}</math> | ||
| + | |style="text-align:right;"|חלקם בי"ז יהיו ל"ח וי"ד חלקים מי"ז | ||
| + | |- | ||
| + | | | ||
| + | ::To know the share of the greater, say: if, when the sum is 17, the share of the greater is 13, how much is his share, when the sum is 60? | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{17}{13}=\frac{60}{Z}}}</math> | ||
| + | |style="text-align:right;"|ולדעת חלקי הגדול אמור אם בעת שהמחובר הוא י"ז יהיה החלק הגדול י"ג בהיות המחובר ס' כמה יהיה חלקו | ||
| + | |- | ||
| + | | | ||
| + | ::Say: 60 times 13 is 780. | ||
| + | |style="text-align:right;"|אמור ס"פ י"ג תש"פ | ||
| + | |- | ||
| + | | | ||
| + | ::Divide it by 17; it is 45 and 15 parts of 17. | ||
| + | ::<math>\scriptstyle{\color{blue}{Z=\frac{60\sdot13}{17}=\frac{780}{17}=45+\frac{15}{17}}}</math> | ||
| + | |style="text-align:right;"|חלקם בי"ז יהיו מ"ה וט"ו חלקים מי"ז | ||
| + | |- | ||
| + | | | ||
| + | ::<span style=color:Green>'''Check:'''</span> The order of the matter is as follows: | ||
| + | |style="text-align:right;"|ויהיה סדר הענין כך | ||
| + | |- | ||
| + | | | ||
| + | ::The smaller says: the sum of [my share], which is 17 and 11 parts [of 17], with half of the middle, which is 19 and 7 parts of 17, and half of the greater, which is 22 and 16 parts of 17, is 60. | ||
| + | |style="text-align:right;"|אומר הקטן אני שאני י"ז וי"א חלקים וחצי האמצעי שהם י"ט וז' חלקים מי"ז וחצי הגדול שהם כ"ב וי"ו חלקים מי"ז מחובר שלשתם ס‫' | ||
| + | |- | ||
| + | |colspan="2"| | ||
| + | ::<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle X+\left[\frac{1}{2}\sdot\left(Y+Z\right)\right]&\scriptstyle=\left(17+\frac{11}{17}\right)+\left[\frac{1}{2}\sdot\left[\left(38+\frac{14}{17}\right)+\left(45+\frac{15}{17}\right)\right]\right]\\&\scriptstyle=\left(17+\frac{11}{17}\right)+\left(19+\frac{7}{17}\right)+\left(22+\frac{16}{17}\right)=60\\\end{align}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | ::The middle says: the sum of [my share], which is 38 and 14 parts of 17, with a third of the smaller, which is 5 and 15 parts of 17, and a third of the greater, which is 15 and 5 parts of 17, is 60. | ||
| + | |style="text-align:right;"|ואומר האמצעי אני שאני ל"ח וי"ד חלקים מי"ז ושליש הקטן שהם ה' וט"ו חלקים מי"ז ושליש הגדול שהם ט"ו וה' חלקים מי"ז מחובר שלשתנו ס‫' | ||
| + | |- | ||
| + | |colspan="2"| | ||
| + | ::<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle Y+\left[\frac{1}{3}\sdot\left(X+Z\right)\right]&\scriptstyle=\left(38+\frac{14}{17}\right)+\left[\frac{1}{3}\sdot\left[\left(17+\frac{11}{17}\right)+\left(45+\frac{15}{17}\right)\right]\right]\\&\scriptstyle=\left(38+\frac{14}{17}\right)+\left(5+\frac{15}{17}\right)+\left(15+\frac{5}{17}\right)=60\\\end{align}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | ::The greater says: the sum of [my share], which is 45 and 15 parts of 17, with a quarter of the smaller, which is 4 and 7 parts of 17, and a quarter of the middle, which is 9 and 12 parts of 17, is 60. | ||
| + | |style="text-align:right;"|ואומר הגדול אני שאני מ"ה וט"ו ‫<ref>88r</ref>חלקים מי"ז ורביע הקטן שהוא ד' וז' חלקים מי"ז ורביע האמצעי שהוא ט' וי"ב חלקים מי"ז מחובר שלושתנו עולה ס‫' | ||
| + | |- | ||
| + | |colspan="2"| | ||
| + | ::<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle Z+\left[\frac{1}{4}\sdot\left(X+Y\right)\right]&\scriptstyle=\left(45+\frac{15}{17}\right)+\left[\frac{1}{4}\sdot\left[\left(17+\frac{11}{17}\right)+\left(38+\frac{14}{17}\right)\right]\right]\\&\scriptstyle=\left(45+\frac{15}{17}\right)+\left(4+\frac{7}{17}\right)+\left(9+\frac{12}{17}\right)=60\\\end{align}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | *Question: Jacob divided [a certain amount] between his three sons. | ||
| + | :The share of Reuven was greater than the share of Shimon and the share of Shimon was greater than the share of Levi. | ||
| + | :Reuven gave his two brothers from his shares as much as their shares. | ||
| + | :Shimon gave his brothers also as much as their shares. | ||
| + | :Levi gave his brothers also as much as their shares. | ||
| + | :Then the shares of the three were equal. | ||
| + | :How much was the [original] share of each? | ||
| + | |style="text-align:right;"|<big>שאלה</big> יעקב חלק לבניו ג' והיה חלק ראובן גדול משל שמעון ושל שמעון גדול משל לוי<br> | ||
| + | נתן ראובן לשני אחיו מחלקו כפי מה שהיה חלקם<br> | ||
| + | ושמעון גם הוא נתן לאחיו כפי חלקם<br> | ||
| + | ולוי גם כן נתן לאחיו כפי חלקם<br> | ||
| + | ואז נמצא חלק שלשתם שוה<br> | ||
| + | כמה היה חלק כל א' מהם | ||
| + | |- | ||
| + | | | ||
| + | ::Say that the share of the first was 4, the share of the second was 7, and the share of the third was 13. We find that at the end their shares were the same: 8 pešuṭim for each. | ||
| + | |style="text-align:right;"|אמור כי חלק הראשון היה ד' וחלק השני ז' וחלק השלישי י"ג ונמצא בסוף חלקם שוה והיה ח' פשוטים לכל א‫' | ||
| + | |- | ||
| + | | | ||
| + | ::For example: Reuven's share was 13, Shimon's share was 7, and Levi's share is 4. | ||
| + | |style="text-align:right;"|כיצד דרך משל חלק ראובן היה י"ג וחלק שמעון ז' וחלק לוי ד‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Reuven starts and gives of his share 7 to Shimon, which is as much as he has, and 4 to Levi; he is left with two. | ||
| + | |style="text-align:right;"|והנה התחיל ראובן ונתן מחלקו לשמעון ז' כפי שהיה לו וללוי ד' ושנים נשאר בידו | ||
| + | |- | ||
| + | |colspan="2" | | ||
| + | ::<math>\scriptstyle{\color{blue}{\begin{cases}\scriptstyle13-7-4=2\\\scriptstyle7+7=14\\\scriptstyle4+4=8\end{cases}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | ::Next, Shimon, who now has 14, gives 8 to Levi, which is as much as he has, and 2 to Reuven, which is as much as he has now. | ||
| + | |style="text-align:right;"|ואח"כ שמעון שעכשיו היה לו י"ד נתן ללוי ח' כפי מה שהיה בידו ולראובן ב' כפי מה שהיה עכשיו בידו | ||
| + | |- | ||
| + | |colspan="2" | | ||
| + | ::<math>\scriptstyle{\color{blue}{\begin{cases}\scriptstyle14-8-2=4\\\scriptstyle8+8=16\\\scriptstyle2+2=4\end{cases}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | ::Then, Levi, who now has 16, gives 4 of them to Reuven, so he has 8, and 4 to Shimon. | ||
| + | |style="text-align:right;"|ואח"כ לוי שעכשיו היה לו י"ו נתן מאלו לראובן ד' ונמצא שיש לו ח' וד' לשמעון | ||
| + | |- | ||
| + | |colspan="2" | | ||
| + | ::<math>\scriptstyle{\color{blue}{\begin{cases}\scriptstyle16-4-4=8\\\scriptstyle4+4=8\\\scriptstyle4+4=8\end{cases}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | ::We find that each has 8 pešuṭim and their shares are the same. | ||
| + | |style="text-align:right;"|ועם זה נמצא שביד כולם יש ח' פשוט' וחלקם שוה | ||
| + | |- | ||
| + | !<span style=color:Green>Buy and Sell Problems</span> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:figs|641|qJg7}}A man said: here are two baskets of figs in each there are 100 figs, the fine are [sold at a rate of] 20 for one pašuṭ, the defective are [sold at a rate of] 30 for one pašuṭ. | ||
| + | :Their total price is 8 pešuṭim and one third. | ||
| + | :One comes to buy and asks: How do you sell [them]? | ||
| + | :He says: I give you [..] 20 of these. | ||
| + | :The buyer answers: give me 20 of these and 20 of these for 2 pešuṭim. | ||
| + | :He sold all for 8 pešuṭim and lost a third of one pašuṭ. | ||
| + | |style="text-align:right;"|אדם א' אמר הנה שני כלכלות של תאנים ובכל א' יש ק' תאנים משל היפות כ' לפשוט והרעות ‫<ref>88v</ref>ל' לפשוט<br> | ||
| + | עולה חשבונם ח' פשוטים ושליש<br> | ||
| + | בא א' לקנות ואמר היאך אתה מוכר<br> | ||
| + | אמר מאלו אתן לך לז' ומאלו כ‫'<br> | ||
| + | השיב הקונה אם כן תן לי מאלו ומאלו כ' בב' פשוטים<br> | ||
| + | ונתן הכל בח' פשוטים והפסיד שליש פשוט{{#annotend:qJg7}} | ||
| + | |- | ||
| + | | | ||
| + | *A man hires a worker for 30 days at a salary of one pašuṭ per day and he has to pay every day, but he only has six coins that are worth a total of 30 pešuṭim. | ||
| + | :Now it is asked what should be the value of the coins, so that he will be able to pay each day? | ||
| + | |style="text-align:right;"|<big>אדם</big> שכר שכיר לל' יום בשכר [...] פשוט לכל יום והיה צריך לפרוע מידי יום ביום ולא היה לו כי אם ששה מטבעות ששוויים בין הכל ל' פשוטים ועכשיו שואלים מה יהיה שווי המטבעות כדי שיוכל לפרוע מידי יום ביומו | ||
| + | |- | ||
| + | | | ||
| + | ::Answer: the value of the coins should be as follows: 1, 2, 3, 8, 12. | ||
| + | |style="text-align:right;"|תשובה שיווי המטבעות צריך להיות כזה א' ב' ג' ד' ח' י"ב | ||
| + | |- | ||
| + | !<span style=color:Green>Ordering Problem</span> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | *A man had a wolf, a goat, and a cabbage, and he had to move them across the water. | ||
| + | :He had nothing but a small boat that could contain only the person and one more thing. | ||
| + | :If he will leave the goat and the cabbage and move the wolf the goat will eat the cabbage. | ||
| + | :If he will move the cabbage and leave the wolf with the goat the wolf will eat the goat. | ||
| + | :Likewise, if he moves one and returns to take the other to move it, while he comes to take it, the first will eat the other. | ||
| + | :How will he do it? | ||
| + | |style="text-align:right;"|אדם היה לו זאב ועז וכרוב והיה לו לעבור מעבר מים ולא היה כי אם ספינה קטנה מאד שאינה מחזיק כי אם האדם ודבר אחד ולא יותר<br> | ||
| + | ואם תניח העז והכרוב ויעביר הזאב העז אכל הכרוב<br> | ||
| + | ואם הכרוב יעביר הכרוב ויניח הזאב אצל העז הזאב יאכל העז<br> | ||
| + | וכמו כן אם יעביר הא' ויחזור ליקח השני להעביר בעוד שיבא ליקח א' יאכל א' חבירו<br> | ||
| + | ואם כן כיצד יעשה | ||
| + | |- | ||
| + | | | ||
| + | :He should move first the goat, leaving the wolf and the cabbage. | ||
| + | |style="text-align:right;"|תחלה יעביר העז ויניח הזאב והכרוב | ||
| + | |- | ||
| + | | | ||
| + | :Then, he should move the cabbage and bring the goat back with him. | ||
| + | |style="text-align:right;"|ואחר יעביר הכרוב ויחזור להעביר העז עמו ‫<ref>89r</ref>למקום הראשון | ||
| + | |- | ||
| + | | | ||
| + | :Then he should move the wolf to the other side and go back to move the goat with him to the other side. | ||
| + | |style="text-align:right;"|ויעביר הזאב לעבר האחר ויחזור להעביר העז עמו לעבר הא' ויעביר הכרוב ויניח העז ואחר יחזור ליקח העז | ||
| + | |- | ||
| + | !<span style=color:Green>Joint Purchase Problem - "If You Give Me"</span> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | *Reuven told Shimon: if you will give me one thousand zehuvim my amount will be equal to your amount of money. | ||
| + | :Shimon answered Reuven: if you will give me one thousand zehuvim my money will be double your amount of money. | ||
| + | :How much money did each of them have?<br> | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle X+1000=Y-1000\\\scriptstyle 2\sdot\left(X-1000\right)=Y+1000\end{cases}</math> | ||
| + | |style="text-align:right;"|ראובן אמר לשמעון אם אתה נותן לי אלף זהובים יעלה הקרן שלי כסכום מעותיך<br> | ||
| + | ושמעון משיב לראובן ואם אתה נותן לי אלף זהובים אני אכפול מעותי לסכום מעותיך<br> | ||
| + | כמה מעות יש לכל אחד | ||
| + | |- | ||
| + | | | ||
| + | ::Answer: Reuven has 5 thousand zehuvim and Shimon has 7 thousand zehuvim. | ||
| + | |style="text-align:right;"|תשובה ראובן יש לו ה' אלפים זהובים ושמעון יש לו ז' אלפים | ||
| + | |- | ||
| + | |colspan="2" | | ||
| + | ::<math>\scriptstyle{\color{blue}{\begin{cases}\scriptstyle X=5000\\\scriptstyle Y=7000\end{cases}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | ::This is the explanation: Reuven asks Shimon for one thousand zehuvim. If Shimon gives him that, Shimon is left with 6 thousand and Reuven, who had 5 thousand at the beginning, now has 6 thousand with the thousand that Shimon gave him. We find that they each have 6 thousand. | ||
| + | ::<math>\scriptstyle{\color{blue}{5000+1000=6000=7000-1000}}</math> | ||
| + | |style="text-align:right;"|וזה פי' ראובן שואל לשמעון אלף זהובים ואם שמעון יתן לו נשאר ביד שמעון ו' אלפים וראובן שהיה לו ה' אלפים תחלה עכשיו עם האלף שנותן לו שמעון יש לו ו' אלפים נמצא שלכל א' יש להם ו' אלפים | ||
| + | |- | ||
| + | | | ||
| + | ::Shimon says to Reuven: "If you give me one thousand zehuvim". We find that Reuven has 4 thousand left and Shimon's amount, which was 7 thousand rises now to 8 thousand, i.e. twice as much as what Reuven is left with. | ||
| + | |style="text-align:right;"|ושמעון אומר לראובן אם אתה תתן לי אלף זהובים נמצא שישאר ביד ראובן ד' אלפים והקרן של שמעון שהיה ז' אלפים נמצא עכשיו עולה לסכום ח' אלפים והיינו הכפל ממה שנשאר ביד ראובן | ||
| + | |- | ||
| + | |colspan="2" | | ||
| + | ::<math>\scriptstyle{\color{blue}{2\sdot\left(5000-1000\right)=2\sdot4000=8000=7000+1000}}</math> | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | == Chapter On Cubic Roots [P1088 2r-v] == | ||
| + | |||
| + | |style="text-align:right;"|והילך שער על דבר מרובע על כל עבריו | ||
| + | |- | ||
| + | | | ||
| + | :*As when you multiply 568 times 568 and this is a square. | ||
| + | ::<math>\scriptstyle{\color{blue}{568\times568}}</math> | ||
| + | |style="text-align:right;"|כגון שתחשוב חוה פעם חוה וזהו רבוע | ||
| + | |- | ||
| + | | | ||
| + | :*As when you say 2 times 2 is 4; like this: | ||
| + | ::<math>\scriptstyle{\color{blue}{2\times2=4}}</math> | ||
| + | |style="text-align:right;"|כמו שתאמר ב פעמים ב' זהו ד' כזה | ||
| + | |- | ||
| + | | | ||
| + | ::If you say: multiply the 4 twice for the width; it is 8 and this is a cube. | ||
| + | ::<math>\scriptstyle{\color{blue}{2\times2\times2=4\times2=8}}</math> | ||
| + | |style="text-align:right;"|ואם תאמר ותחשוב הד' חתיכות גם ב' פעמים לעובין ויהיו ח' זהו רבוע על כל עבריו | ||
| + | |- | ||
| + | | | ||
| + | ::2 times 2 is 4. | ||
| + | |style="text-align:right;"|ב' פעם ב' זהו ד‫' | ||
| + | |- | ||
| + | | | ||
| + | ::2 times 4 is 8. | ||
| + | |style="text-align:right;"|ב' פעמים ד' זהו ח' כו‫' | ||
| + | |- | ||
| + | | | ||
| + | :*When you multiply 464 times 464 the result is 215296. | ||
| + | ::<math>\scriptstyle{\color{blue}{464\times464=215296}}</math> | ||
| + | |style="text-align:right;"|כשתחשוב דוד פעם דוד עולה וטבהאב | ||
| + | |- | ||
| + | | | ||
| + | ::Say: 464 times 215296; the result is 99897344. | ||
| + | ::<math>\scriptstyle{\color{blue}{464\times464\times464=464\times215296=99897344}}</math> | ||
| + | |style="text-align:right;"|תאמר דוד פעם וטבהאב ועולה דדגזטחטט כו‫' | ||
| + | |- | ||
| + | | | ||
| + | *Example: the total of the cubic product is like this: | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt[3]{149721291}}}</math> | ||
| + | |style="text-align:right;"|דומיון הנה הכלל העולה מן החשבון המרובע לכל צדדיו הוא כזה | ||
| + | |- | ||
| + | | | ||
| + | :149721291 | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |style="text-align:right;"|א||ד||ט||ז||ב||א||ב||ט||א | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Always count fours, as 149, 9721, 1291 and from the digits less that four you can find the number. | ||
| + | |style="text-align:right;"|ותמנה לעולם ברביעיות כגון אטבא אבזט טדא ומה שלא עולה לרבעיות מאותן אותיות תוכל להבין החשבון | ||
| + | |- | ||
| + | | | ||
| + | ::Now, in the written total, 149 are 3 digits, so say: which cube is in these three digits 149? It is no more than 5, because 5 times 5 is 25 and 5 times 25 is 125. | ||
| + | |style="text-align:right;"|עתה בכלל הנרשם טדא ג' אותיות ותאמר מה הריבוע המרובע על כל עבריו <s>אטדא</s> בזה ג' אותיות טדא ע"כ לא יותר מן ה' כי ה' פעמי' ה' זהו ה"ב ה' פעמים ה"ב זהו הבא | ||
| + | |- | ||
| + | | | ||
| + | ::Subtract 125 from 149; 24 remains, like this: | ||
| + | |style="text-align:right;"|ועתה הסר הבא מן טדא ונשאר ד"ב ויהא כזה | ||
| + | |- | ||
| + | | | ||
| + | ::Write 5 beneath the 4 and above the 4, like this: | ||
| + | |style="text-align:right;"|ותכתוב ה' תחת הד' ועל הד' כזה | ||
| + | |- | ||
| + | | | ||
| + | :{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | | ||5|| || || || || || | ||
| + | |- | ||
| + | |2||4||7||2||1||2||9||1 | ||
| + | |- | ||
| + | | ||5|| || || || || || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | | ||ה|| || || || || || | ||
| + | |- | ||
| + | |ב||ד||ז||ב||א||ב||ט||א | ||
| + | |- | ||
| + | | ||ה|| || || || || || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Triple the number found, i.e. 5, and shift it three ranks back, i.e. from 5; it is 15. | ||
| + | |style="text-align:right;"|ומעתה תשלש האות הנמצא ר"ל ה' ונסוג שלש מעלות לאחריך דהיינו מן ה' יהיה ה"א | ||
| + | |- | ||
| + | | | ||
| + | ::Write 5 beneath 2 and 1 beneath 7, like this: | ||
| + | |style="text-align:right;"|ותכתוב ה' תחת ב' וא' תחת ז' כזה | ||
| + | |- | ||
| + | | | ||
| + | :{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | | || || ||5|| || || || | ||
| + | |- | ||
| + | |2||4||7||2||1||2||9||1 | ||
| + | |- | ||
| + | | || ||1||5|| || || || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | | || || ||ה|| || || || | ||
| + | |- | ||
| + | |ב||ד||ז||ב||א||ב||ט||א | ||
| + | |- | ||
| + | | || ||א||ה|| || || || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | |colspan="2" | | ||
| + | :{| | ||
| + | |- | ||
| + | | ||rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{Purple}{149-{\color{blue}{5}}^3}}=149-125={\color{blue}{24}}}</math>|| <span style="color:#0000FF>5</span>      ||rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{Purple}{3\times5}}={\color{blue}{15}}}</math>||   5     | ||
| + | |- | ||
| + | |<span style="color:Purple">149</span>721291||<span style="color:#0000FF>24</span>721291||24721291 | ||
| + | |- | ||
| + | | || <span style="color:Purple">5</span>      ||  <span style="color:#0000FF">15</span>     | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Now, see which digit can be added to the digit 5, from which this total number written is generated and check it: add to it a digit that you think of as I will teach you, so that the last digit of the resulting number will correspond to the last digit of the number we are dealing with, or is approximately the same as the last digit. | ||
| + | |style="text-align:right;"|ועתה תעיין מה אות {{#annot:term|178|RBW2}}מצורף ל{{#annotend:RBW2}}אות ה' שממנה בא זה החשבון הכלל הנרשם ואז תנסה ותצרף אליה אות שאם תחשוב כאשר אשכילך שיהא מכוון אות האחרון מן חשבון העולה לאות אחרון מן חשבון אשר אנו מתעסקים בו או בקרוב דומה לאות אחרון | ||
| + | |- | ||
| + | | | ||
| + | ::Here is the procedure you apply: take 3 and write it above the 1, one rank before the 5 that stands above the 2. Like this: | ||
| + | |style="text-align:right;"|ועתה הילך הדרך תלך בו תקח ג' וכתוב למעלה על הא' <s>אחר</s> לפני הה' העומדת על הב' כזה | ||
| + | |- | ||
| + | | | ||
| + | :{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | | || || ||5||3|| || || | ||
| + | |- | ||
| + | |2||4||7||2||1||2||9||1 | ||
| + | |- | ||
| + | | || ||1||5|| || || || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | | || || ||ה||ג|| || || | ||
| + | |- | ||
| + | |ב||ד||ז||ב||א||ב||ט||א | ||
| + | |- | ||
| + | | || ||א||ה|| || || || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Now, check if it is right: multiply the upper 53 by the bottom 15; the result is 795. | ||
| + | |style="text-align:right;"|ועתה נסה אם אמת הדבר {{#annot:|185|WCLM}}תצרף{{#annotend:WCLM}} ג"ה של מעלה עם ה"א של מטה ויעלה הטז | ||
| + | |- | ||
| + | | | ||
| + | ::Multiply 3 by 795: 3 times 7, 3 times 9, 3 times 5; the result is like this: | ||
| + | |style="text-align:right;"|ועתה <s>תאמר</s> תצרף ג' העולה עם הט"ז ג' פעמים ז' ג' פעמי' ט' ג' פעמי' ה' כו ויעלה כזה | ||
| + | |- | ||
| + | | | ||
| + | :{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |2||3||8||5 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ב||ג||ח||ה | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Then, add to this number [the cube of] 3, which is 27. Write 27 on 2385 and do like this: write the first digit of 27, i.e. the 7, before 2385 and write the second digit, i.e. 2, above 5, like this: | ||
| + | |style="text-align:right;"|ואז תוסיף <s>עליו</s> על זה החשבון ג' פעמי' ג' על כל עבריו זהו ז"ב אותו ז"ב שים על החג"ב וככה תעשה אות ראשון מן ז"ב ר"ל הז' כתוב <s>קו</s> לפני החג"ב ואות השיני דהיינו ב' שים על ה' כזה | ||
| + | |- | ||
| + | | | ||
| + | :{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |2||3||8||7||7 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ב||ג||ח||ז||ז | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | |colspan="2" | | ||
| + | |||
| + | :{| | ||
| + | |- | ||
| + | |   5<span style="color:#0000FF>3</span>   ||rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{blue}{3}}\times{\color{Purple}{53\times15}}={\color{blue}{2385}}}</math>|| ||rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{Purple}{23850+}}{\color{Purple}{3^3}}=23850+27=238{\color{blue}{77}}}</math>|| | ||
| + | |- | ||
| + | |24721291|| || | ||
| + | |- | ||
| + | |  <span style="color:Purple">15</span>    ||<span style="color:#0000FF>2385</span>||238<span style="color:#0000FF>77</span> | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Write these 5 digits corresponding to the 5 digits of the written total like this: | ||
| + | |style="text-align:right;"|וזה ה' אותיות תשים נגד ה' אותיות הכלל הנרשם דהוא כזה | ||
| + | |- | ||
| + | | | ||
| + | :{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |2||4||7||2||1||2||9||1 | ||
| + | |- | ||
| + | |2||3||8||7||7|| || || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ב||ד||ז||ב||א||ב||ט||א | ||
| + | |- | ||
| + | |ב||ג||ח||ז||ז|| || || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Subtract the bottom from the upper; the remainder is like this: | ||
| + | |style="text-align:right;"|ותחסר כלל התחתון מן העליון ונשאר כזה | ||
| + | |- | ||
| + | | | ||
| + | :{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |8||4||4||2||9||1 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ח||ד||ד||ב||ט||א | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | |colspan="2" | | ||
| + | :{| | ||
| + | |- | ||
| + | | ||rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{Purple}{24721-23877}}={\color{blue}{844}}}</math>|| | ||
| + | |- | ||
| + | |<span style="color:Purple">24721</span>291||<span style="color:#0000FF>844</span>291 | ||
| + | |- | ||
| + | |<span style="color:Purple">23877</span>    || | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Now, the diagram of the number is like this: | ||
| + | |style="text-align:right;"|ועתה יהיה החשבון צורתו כזה | ||
| + | |- | ||
| + | | | ||
| + | :{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | | ||5||3|| || || | ||
| + | |- | ||
| + | |8||4||4||2||9||1 | ||
| + | |- | ||
| + | |1||5|| || || || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | | ||ה||ג|| || || | ||
| + | |- | ||
| + | |ח||ד||ד||ב||ט||א | ||
| + | |- | ||
| + | |א||ה|| || || || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::We shift [5] with the 1, three ranks backwards. | ||
| + | |style="text-align:right;"|ועתה נסוג ג' עם הא' ג' מעלות לאחריך | ||
| + | |- | ||
| + | | | ||
| + | ::We shift 3 backwards three ranks as well. Triple [it]; it is 9. The diagram is like this: | ||
| + | |style="text-align:right;"|ונסוג ג' ג"כ לאחריך ג' מעלות ותשלש ויהיה ט' וצורתו כזה | ||
| + | |- | ||
| + | | | ||
| + | :{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |8||4||4||2||9||1 | ||
| + | |- | ||
| + | | || || ||1||5||9 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ח||ד||ד||ב||ט||א | ||
| + | |- | ||
| + | | || || ||א||ה||ט | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | |colspan="2" | | ||
| + | ::{| | ||
| + | |- | ||
| + | | 5<span style="color:Purple">3</span>   ||rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{Purple}{3\times3}}={\color{blue}{9}}}</math>|| | ||
| + | |- | ||
| + | |844291||844291 | ||
| + | |- | ||
| + | |15    ||   15<span style="color:#0000FF>9</span> | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Now say: what is the first digit? It is 1, because when 1 is the first digit of the total, the unit of the result is always 1, and if the first digit is 8, the units are 2. | ||
| + | |style="text-align:right;"|ועתה תאמר מה היה אות ראשון ע"כ היה א' כי לעולם כשיהיה א' אות ראשון מן הכלל העולה היה הפרט א' ואם אות ראשון ח' אז היה הפרט ב‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Multiply 531 by 159; the result is 84429. | ||
| + | |style="text-align:right;"|ועתה תחשב אג"ה עם טה"א ויעלה טבדדח | ||
| + | |- | ||
| + | |colspan="2" | | ||
| + | :{| | ||
| + | |- | ||
| + | |<math>\scriptstyle\xrightarrow{{\color{Purple}{53{\color{blue}{1}}\times159}}={\color{blue}{84429}}}</math>||<span style="color:#0000FF>84429</span> | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Multiply 1 by each digit of 844291 and write 1 times 1 first; it is like this: | ||
| + | |style="text-align:right;"|ועתה תצרף הא' ג"כ עם כל אות אטבדד"ח ותשים לפניו א' פעמים א' ויהיה כזה | ||
| + | |- | ||
| + | | | ||
| + | :{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |8||4||4||2||9||1 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ח||ד||ד||ב||ט||א | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | |colspan="2" | | ||
| + | :{| | ||
| + | |- | ||
| + | |<math>\scriptstyle\xrightarrow{{\color{Purple}{844290+1}}={\color{blue}{844291}}}</math>||84429<span style="color:#0000FF>1</span> | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::We find that the product of 531 times 159 with one and the total written above match and when they match, the calculation is correct, but if you have even one digit left, you can understand that it is incorrect. | ||
| + | |style="text-align:right;"|נמצא שהם מכוונים זה העולה מן אג"ה פעמים טה"א אחד צרופך והכלל הנרשם למעלה ולעולם כשהם מכוונים אז החשבון אמת ואם יוותר לך אפי' אות אחת תוכל להבין שלא [נאות] | ||
| + | |- | ||
| + | | | ||
| + | *Another example: the total result is 99897344. | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt[3]{99897344}}}</math> | ||
| + | |style="text-align:right;"|דומיון אחר הכלל העולה ד ד ג ז ט ח ט ט | ||
| + | |- | ||
| + | | | ||
| + | ::We start from the first digit 9, because it is the [eighth] digit. We take the cube from 99; it is 4, because you cannot take more. | ||
| + | |style="text-align:right;"|נתחיל מאות ט' הראשונה כי היא אות רביעית ונקח מן ט"ט הריבוע והוא ע"כ ד' כי לא תוכל ליקח יותר והוא ד' פעמים ד' עלה ד"ו | ||
| + | |- | ||
| + | | | ||
| + | ::It is 4 times 4; the result is 64. Subtract 64 from 99; 35 remains. | ||
| + | |style="text-align:right;"|חסר ד"ו מן ט"ט ונשאר ה"ג | ||
| + | |- | ||
| + | | | ||
| + | ::Write 4 beneath and above the 9. | ||
| + | |style="text-align:right;"|אותו ד' תכתוב למטה תחת הט' ולמעלה | ||
| + | |- | ||
| + | | | ||
| + | ::Shift 4 three ranks back and triple it; it is 12. | ||
| + | |style="text-align:right;"|נסוג ד' ג' מעלות ותשלש לאחריך ויהיה ב"א | ||
| + | |- | ||
| + | | | ||
| + | ::Write 2 beneath the 9 and 1 beneath 8, like this: | ||
| + | |style="text-align:right;"|תכתוב ב' תחת הט' וא' תחת ח' כזה | ||
| + | |- | ||
| + | | | ||
| + | :{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | | || ||4||6|| || || || | ||
| + | |- | ||
| + | |3||5||8||9||7||3||4||4 | ||
| + | |- | ||
| + | | || ||1||2|| || || || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | | || ||ד||ו|| || || || | ||
| + | |- | ||
| + | |ג||ה||ח||ט||ז||ג||ד||ד | ||
| + | |- | ||
| + | | || ||א||ב|| || || || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | |colspan="2" | | ||
| + | :{| | ||
| + | |- | ||
| + | | ||rowspan="3"|<math>\scriptstyle\xrightarrow{\scriptstyle\begin{cases}\scriptstyle{\color{Purple}{99-{\color{blue}{4}}^3}}=99-64={\color{blue}{35}}\\\scriptstyle{\color{Purple}{3\times4=}}{\color{blue}{12}}\end{cases}}</math>||  <span style="color:#0000FF>46</span>     | ||
| + | |- | ||
| + | |<span style="color:Purple">99</span>897344||<span style="color:#0000FF>35</span>897344 | ||
| + | |- | ||
| + | | ||  <span style="color:#0000FF">12</span>     | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Then, add to the 4 a number that you think is appropriate; it is 6. | ||
| + | |style="text-align:right;"|ואח' תצרף אל הד' <s>ד'</s> מנין השוה בדעתך וזהו ו‫' | ||
| + | |- | ||
| + | | | ||
| + | ::If you add 5 to it, the root will not match the total. | ||
| + | |style="text-align:right;"|ואם תצרף אליו ה' אז אינו מכוון פרט העולה לחשבון הכלל | ||
| + | |- | ||
| + | | | ||
| + | ::Sometimes it cannot really match, but you approximate with a digit that is close to the latter digit. | ||
| + | |style="text-align:right;"|ולפעמים לפי האמת אינו מכוון אך תדקדק על אות הסמוך לאות אחרון שהוא דומה קצת | ||
| + | |- | ||
| + | | | ||
| + | ::Now, take 6, add it to 4, and say: 46 times 12; the result is 552. | ||
| + | |style="text-align:right;"|ועתה קח ו' ותצרף לד' ותאמר ו"ד פעמי' ב"א ויעלה בה"ה | ||
| + | |- | ||
| + | | | ||
| + | ::Multiply 6 by 552; the result is like this: | ||
| + | |style="text-align:right;"|תצרף ו' עם בה"ה ויעלה כזה | ||
| + | |- | ||
| + | | | ||
| + | :{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |3||3||1||2 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ג||ג||א||ב | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |||
| + | |- | ||
| + | |colspan="2" | | ||
| + | :{| | ||
| + | |- | ||
| + | |<math>\scriptstyle\xrightarrow{{\color{blue}{6}}\times{\color{Purple}{46\times12}}=6\sdot552={\color{blue}{3312}}}</math>||<span style="color:#0000FF>3312</span> | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Add to it 6 times 6; it is like this: | ||
| + | |style="text-align:right;"|ושים עליו ו' פעמים ו' ויהיה כזה | ||
| + | |- | ||
| + | | | ||
| + | :{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |3||3||3||3||6 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ג||ג||ג||ג||ו | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | |colspan="2" | | ||
| + | :{| | ||
| + | |- | ||
| + | |<math>\scriptstyle\xrightarrow{{\color{Purple}{33120+}}{\color{Purple}{6^3}}=33{\color{blue}{336}}}</math>||33<span style="color:#0000FF>336</span> | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Subtract the result from the five digits of the written number; it is according to this diagram after the subtraction: | ||
| + | |style="text-align:right;"|תחסר זה העולה מן ה' אותיות החשבון הנרשם ויהיה אחר חסרונך כזה ועתה צורתו כזה | ||
| + | |- | ||
| + | | | ||
| + | :{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | | || ||4||6|| || || | ||
| + | |- | ||
| + | |2||5||6||1||3||4||4 | ||
| + | |- | ||
| + | | ||1||2|| || || || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | | || ||ד||ו|| || || | ||
| + | |- | ||
| + | |ב||ה||ו||א||ג||ד||ד | ||
| + | |- | ||
| + | | ||א||ב|| || || || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | |colspan="2" | | ||
| + | :{| | ||
| + | |- | ||
| + | |rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{Purple}{35897-33336=}}{\color{blue}{2561}}}</math>||  46    | ||
| + | |- | ||
| + | |<span style="color:#0000FF>2561</span>344 | ||
| + | |- | ||
| + | | 12     | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Now, shift 6 three ranks back and triple it; and 12 three ranks back as well. It is like this: | ||
| + | |style="text-align:right;"|ועתה נסוג עם ו' העולה ג' מעלות לאחריך ותשלש וב"א ג"כ ג' מעלות לאחריך ויהיה כזה | ||
| + | |- | ||
| + | | | ||
| + | :{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | | || || || ||4||6|| | ||
| + | |- | ||
| + | |2||5||6||1||3||4||4 | ||
| + | |- | ||
| + | | || || || ||1||3||8 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | | || || || ||ד||ו|| | ||
| + | |- | ||
| + | |ב||ה||ו||א||ג||ד||ד | ||
| + | |- | ||
| + | | || || || ||א||ג||ח | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | |colspan="2" | | ||
| + | :{| | ||
| + | |- | ||
| + | |rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{Purple}{120+\left(3\times6\right)=1}}{\color{blue}{38}}}</math>||    46  | ||
| + | |- | ||
| + | |2561344 | ||
| + | |- | ||
| + | |    1<span style="color:#0000FF>38</span> | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::What is the first digit? 4. | ||
| + | |style="text-align:right;"|ועתה מה היה אות ראשון ע"כ ד‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Multiply 464 by 138; the result is this. Add to it 4 times 4; the result is exactly the same as the total. | ||
| + | |style="text-align:right;"|ותחבר דוד עם חג"א ויעלה כו' ושים עליו ד' פעמי' ד' ויעלה הפרט אות באות כגון הכלל ודוק | ||
| + | |- | ||
| + | |colspan="2" | | ||
| + | :{| | ||
| + | |- | ||
| + | |<math>\scriptstyle\xrightarrow{{\color{Purple}{\left[\left[4\times\left(464\times138\right)\right]\sdot10\right]+4^3}}={\color{blue}{2561344}}}</math>||<span style="color:#0000FF>2561344</span> | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | == Here is a Chapter, Called the Chapter on Square Roots [P1088 6v-7v] == | ||
| + | |||
| + | |style="text-align:right;"|הילך שער ונקר' שער השורש | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ושייך על דבר כשחשבת ריבוע | ||
| + | |- | ||
| + | | | ||
| + | :*As 568 times 568 | ||
| + | ::<math>\scriptstyle{\color{blue}{568\times568}}</math> | ||
| + | |style="text-align:right;"|כגו' חו"ה פעם חו"ה | ||
| + | |- | ||
| + | | | ||
| + | :*Or, 257 times 257 | ||
| + | ::<math>\scriptstyle{\color{blue}{257\times257}}</math> | ||
| + | |style="text-align:right;"|או זה"ב פעם זה"ב | ||
| + | |- | ||
| + | | | ||
| + | :When you first see the product even though you do not know the root first. | ||
| + | |style="text-align:right;"|שתראה מה תחילת החשבון אע"ג שלא ידעת בתחילה הפרט | ||
| + | |- | ||
| + | | | ||
| + | *Now, I will write an example for you: I multiplied a thing and the resulting total is like this: 215296. | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{215296}}}</math> | ||
| + | |style="text-align:right;"|ועת' אכתו' דומיון חשבתי דבר ועולה ממנה כזה הוה הכלל<br> | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |style="text-align:right;"|ב||א||ה||ב||ט||ו | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | :See how much is the original number it resulted from. | ||
| + | |style="text-align:right;"|וראה מה היה מתחילה הפרט שעולה ממנ‫' | ||
| + | |- | ||
| + | | | ||
| + | :Now, I will teach you and "''let a wise man, and he will become wiser… and he will increase in learning''" [Proverbs 9, 9]. | ||
| + | |style="text-align:right;"|ועתה אשכילך ו''{{#annot:Pr9-9|494|zAtD}}תן לחכם ויחכם עוד ויסף לקח{{#annotend:zAtD}}''‫<ref group=note>משלי ט, ט</ref> | ||
| + | |- | ||
| + | | | ||
| + | :Take the total and I will show you the root [lit. thing]: | ||
| + | |style="text-align:right;"|קח הכלל לפניך ואראה לך דבר | ||
| + | |- | ||
| + | | | ||
| + | :Always start from the digit [?] | ||
| + | |style="text-align:right;"|לעולם תתחיל מן האו' שלא מכוון | ||
| + | |- | ||
| + | | | ||
| + | :For example: if there are 6 digits, start from the fifth digit. | ||
| + | |style="text-align:right;"|כגו' <s>אם ו' ז' או ט'</s> אם האותיות ו' אז תתחיל באות ה‫' | ||
| + | |- | ||
| + | | | ||
| + | :If there are 8 digits, start from seventh digit. | ||
| + | |style="text-align:right;"|ואם האותיו' ח' אז תתחיל באות ז‫' | ||
| + | |- | ||
| + | | | ||
| + | :And so on. | ||
| + | |style="text-align:right;"|וכן לעולם | ||
| + | |- | ||
| + | | | ||
| + | :If there are 7 digits, start from the last digit. | ||
| + | |style="text-align:right;"|ואם האותיו' ז' אז תתחיל באות האחרון | ||
| + | |- | ||
| + | | | ||
| + | ::Now, I see that the total number has six digits, 215296, so start from the fifth digit, which is 1, and say: what square is found in 21, meaning what digit can you take as many times as itself? | ||
| + | |style="text-align:right;"|ועתה אראה חשב' הכלל ו' אותיות ו"טב"הא"ב אז תתחיל באו' ה' וזהו א' ותאמ' מה הריבוע בא"ב פי' מה אות אתה יכול ליקח זה פעם זה | ||
| + | |- | ||
| + | | | ||
| + | ::You cannot take 5 times 5 from 21, because 5 times 5 is 25, but you only have 21. | ||
| + | |style="text-align:right;"|אי אתה יכול ליקח ה' פעם ה' מן א"ב כי ה' פעם ה' זהו כ"ה והשו' אין לך אלא כ"א | ||
| + | |- | ||
| + | | | ||
| + | ::But, you can take 4 times 4, because 4 times 4 is 16 and you still have 5 left. So, the result is the digit 4, because the square contained in 21 is 4 times 4. | ||
| + | |style="text-align:right;"|אלא אתה יכול [לומ'] ליקח ד' פעם ד' כי ד' פעם ד' זהו ו"א פי' י"ו ועוד נשאר לך ה' ואות' ד' העולה כי הריבוע בא"ב זהו ד' פעם ד‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Write the 4 above the 1 of 215296 and beneath the 1. | ||
| + | |style="text-align:right;"|ואות' ד' תכתוב על הא' מן ו'ט'ב'ה'א'ב' ותחת הא‫' <s>וצורת</s> | ||
| + | |- | ||
| + | | | ||
| + | ::Erase the 21 of 215296 and write 5 instead, because 5 remains from 4 times 4. | ||
| + | |style="text-align:right;"|ותמחק הא"ב מן וטבהא"ב ותכתוב במקומ' ה' כי מן ד' פעם ד' נשאר ה‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Write the 4 above the 5 and beneath the 5. Here is the diagram: | ||
| + | |style="text-align:right;"|והד' תכתו' למעלה מן הה' ולמט' מן הה' והילך צורתו | ||
| + | |- | ||
| + | | | ||
| + | :{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |4|| || || || | ||
| + | |- | ||
| + | |5||5||2||9||6 | ||
| + | |- | ||
| + | |4|| || || || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ד|| || || || | ||
| + | |- | ||
| + | |ה||ה||ב||ט||ו | ||
| + | |- | ||
| + | |ד|| || || || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Double the 4 of the square [root] and shift the 8 backwards, beneath the second 5. Shift backwards also the 4 that stands above the 5 above the second 5, but do not double it. The diagram is like this: | ||
| + | |style="text-align:right;"|ואז תכפול הד' הריבוע העומד [ותמשוך] הח' לאחריה תחת ה' השנייה ויהא ח' והד' העומדת על הה' תמשוך ג"כ לאחריה על ה' השניי' אבל לא תכפול אות' וצורתו כזה | ||
| + | |- | ||
| + | | | ||
| + | :{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | | ||4|| || || | ||
| + | |- | ||
| + | |5||5||2||9||6 | ||
| + | |- | ||
| + | | ||8|| || || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | | ||ד|| || || | ||
| + | |- | ||
| + | |ה||ה||ב||ט||ו | ||
| + | |- | ||
| + | | ||ח|| || || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | |colspan="2" | | ||
| + | :{| | ||
| + | |- | ||
| + | | ||rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{Purple}{21-{\color{blue}{4}}^2}}=21-16={\color{blue}{5}}}</math>||<span style="color:#0000FF>4</span>    ||rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{Purple}{2\times4}}={\color{blue}{8}}}</math>|| 4    | ||
| + | |- | ||
| + | |<span style="color:Purple>21</span>5296||<span style="color:#0000FF>5</span>5296||55296 | ||
| + | |- | ||
| + | | ||<span style="color:Purple">4</span>    || <span style="color:#0000FF">8</span>    | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Then, say: how many times is 8 in 55? It is 6 times in it. | ||
| + | |style="text-align:right;"|ואז תאמר כמה פעם ח' בה"ה פי' בנ"ה והוה בה ו' פעמים | ||
| + | |- | ||
| + | | | ||
| + | ::Write 6 above the 2 and beneath the 2, like this: | ||
| + | |style="text-align:right;"|אות' ו' תכתוב למעלה מן הב' ולמטה מן הב' כזה | ||
| + | |- | ||
| + | | | ||
| + | :{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | | ||4||6|| || | ||
| + | |- | ||
| + | |5||5||2||9||6 | ||
| + | |- | ||
| + | | ||8||6|| || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | | ||ד||ו|| || | ||
| + | |- | ||
| + | |ה||ה||ב||ט||ו | ||
| + | |- | ||
| + | | ||ח||ו|| || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::7 still remains from 55. Erase the second 5 and write the 7 instead. The diagram is like this: | ||
| + | |style="text-align:right;"|ועוד נשאר ז' וז' מן ה"ה ותמחוק השני ה' ותכתו' במקומ' הז' ויהא צורתו כזה | ||
| + | |- | ||
| + | | | ||
| + | :{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |4||6|| || | ||
| + | |- | ||
| + | |7||2||9||6 | ||
| + | |- | ||
| + | |8||6|| || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ד||ו|| || | ||
| + | |- | ||
| + | |ז||ב||ט||ו | ||
| + | |- | ||
| + | |ח||ו|| || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Take also the 6 resulting from the two 5s, which stands above the 2 and beneath the 2. 6 times 6 is 36. Take 36 from 72: take forty from the 70. 2 still remains. Know that you take forty, but you only need 36. So take the 4 from the 40 and write it above the 2 before the 7. The diagram is like this: | ||
| + | |style="text-align:right;"|והו' העולה מן השני ה' העומדת על הב' ולמט' מן הב' תקח אות' נמי ו' פעמי' ו' פעם ו' זהו ו"ג פי' ל"ו וקח הו"ג מן הב"ז קח ארבעי' מן הע' כי הב"ז הוה ע"ב ועוד נשאר ב' ודע שלקחת ארבעי' ולא אתה צרך אל צריך אלא ל"ו אז קח הד' מן המ' ושים אות' על הב' שלפני הז' ויהא צורתו כזה | ||
| + | |- | ||
| + | | | ||
| + | :{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |4||6|| || | ||
| + | |- | ||
| + | |3||6||9||6 | ||
| + | |- | ||
| + | |8||6|| || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ד||ו|| || | ||
| + | |- | ||
| + | |ג||ו||ט||ו | ||
| + | |- | ||
| + | |ח||ו|| || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | |colspan="2" | | ||
| + | :{| | ||
| + | |- | ||
| + | | 4<span style="color:#0000FF>6</span>  ||rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{Purple}{55-\left({\color{blue}{6}}\times8\right)=}}{\color{blue}{7}}}</math>||46  ||rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{Purple}{72-6^2}}=72-36={\color{blue}{36}}}</math>||46   | ||
| + | |- | ||
| + | |<span style="color:Purple>55</span>296||<span style="color:#0000FF>7</span>296||<span style="color:#0000FF>36</span>96 | ||
| + | |- | ||
| + | | 8<span style="color:#0000FF>6</span>  ||8<span style="color:Purple>6</span>  ||86   | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Now, double the 6 as well; it is 12. Write the 12 backwards and the 8 backwards too. Do as follows: write the 2 beneath the 9 and write the 1 above the 8; it is 9. Write the upper 6 backwards as well, but do not double it. Write it above the 9 and shift the upper 4 also backwards, above the 6 corresponding to the 9, like this: | ||
| + | |style="text-align:right;"|ועתה תכפול הו' ג"כ לאחריה ויהא י"ב ואז תכתו' הכי שים הב"א לאחריה והח' נמי לאחרי' ואז תעשה הכי שים הב' תחת הט' והא' שים על הח' ויהיה ט' ותשים נמי למפריע הו' העליונ' ולא תכפול ושי' אות' על הט' והד' העליונ' תמשוך נמי לאחריה על הו' נגד הט' כזה | ||
| + | |- | ||
| + | | | ||
| + | :{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | | ||4||6|| | ||
| + | |- | ||
| + | |3||6||9||6 | ||
| + | |- | ||
| + | | ||9||2|| | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | | ||ד||ו|| | ||
| + | |- | ||
| + | |ג||ו||ט||ו | ||
| + | |- | ||
| + | | ||ט||ב|| | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Then, say: how many times is 9 in 36? It is 4 times in it and you have nothing left. | ||
| + | |style="text-align:right;"|ואז תאמ' כמה פעמים ט' בו"ג פי' בל"ו והוה בו ד' פעמים ולא נשארי' לך מאומה | ||
| + | |- | ||
| + | | | ||
| + | ::Write 4 above the first 6 and beneath the 6, and erase the 36 above and the 9 below, like this: | ||
| + | |style="text-align:right;"|ואות' ד' תכתו' על הו' הראשונ' ותחת הו' ותמחק הו"ג של מעלה והט' של מטה וצורת' כזה | ||
| + | |- | ||
| + | | | ||
| + | :{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |4||6||4 | ||
| + | |- | ||
| + | | ||9||6 | ||
| + | |- | ||
| + | | ||2||4 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ד||ו||ד | ||
| + | |- | ||
| + | | ||ט||ו | ||
| + | |- | ||
| + | | ||ב||ד | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Next, take the 2 also 4 times from the 9; you have 1 left. | ||
| + | |style="text-align:right;"|ואז קח הב' נמי ד' פעמים מן הט' ועוד נשאר לך א‫' | ||
| + | |- | ||
| + | | | ||
| + | ::We erase the 9. Write 1 instead. | ||
| + | |style="text-align:right;"|ונמח' הט' ותכתוב במקו' א‫' | ||
| + | |- | ||
| + | | | ||
| + | ::We erase the bottom 2, because we took it also 4 times. There is still a remainder, like this: | ||
| + | |style="text-align:right;"|ונמח' הב' תחתונ' כי לקחנו נמי ד' פעמי' ועוד נשאר כזה | ||
| + | |- | ||
| + | | | ||
| + | :{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |4||6||4 | ||
| + | |- | ||
| + | | ||1||6 | ||
| + | |- | ||
| + | | || ||4 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ד||ו||ד | ||
| + | |- | ||
| + | | ||א||ו | ||
| + | |- | ||
| + | | || ||ד | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | |colspan="2" | | ||
| + | :{| | ||
| + | |- | ||
| + | |rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{Purple}{80+\left(2\times6\right)=80+12=}}{\color{blue}{92}}}</math>|| 46 ||rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{Purple}{36-\left({\color{blue}{4}}\times9\right)=36-36=0}}}</math>||46<span style="color:#0000FF>4</span>||rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{Purple}{9-\left(4\times2\right)=}}{\color{blue}{1}}}</math>||464 | ||
| + | |- | ||
| + | |<span style="color:Purple>36</span>96|| 96|| <span style="color:#0000FF>1</span>6 | ||
| + | |- | ||
| + | | <span style="color:#0000FF>92</span> || 24||  4 | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Take the 4 also 4 times from 16; you have nothing left. | ||
| + | |style="text-align:right;"|ואז קח הד' נמי ד' פעמי' מן ו"א פי' מן י"ו ואז לא נשאר לך מאומה | ||
| + | |- | ||
| + | | | ||
| + | ::You find that the original factor was 464 times 464 and the resulting total is 215296. | ||
| + | |style="text-align:right;"|נמצא שהפרט היה בתחילה דו"ד פעם דו"ד ועולה הכלל ו"טב"הא"ב | ||
| + | |- | ||
| + | |colspan="2" | | ||
| + | :{| | ||
| + | |- | ||
| + | |<math>\scriptstyle\xrightarrow{{\color{Purple}{16-4^2}}=16-16={\color{blue}{0}}}</math>||<span style="color:#0000FF>464×464=215296</span> | ||
| + | |} | ||
| + | |- | ||
| + | |Always know that when you do it this way and you have nothing left, then the result you received is the original number. | ||
| + | |style="text-align:right;"|ודע לעולם כשתעשה בדרך זה ולא נשאר לך מאומ' אז מה שעולה בידך זה היה תחילת החשבון | ||
| + | |- | ||
| + | | | ||
| + | *I will show you another example: the total number is like this: | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{97344}}}</math> | ||
| + | |style="text-align:right;"|ועוד אראה לך דומיון החשבון עולה כזה | ||
| + | |- | ||
| + | | | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |style="text-align:right;"|ט||ז||ג||ד||ד | ||
| + | |} | ||
| + | |- | ||
| + | |colspan="2" | | ||
| + | :{| | ||
| + | |- | ||
| + | |<span style="color:#0000FF>3</span>    ||rowspan="3"|<math>\scriptstyle\xrightarrow{\scriptstyle\begin{cases}\scriptstyle{\color{Purple}{9-{\color{blue}{3}}^2=9-9-0}}\\\scriptstyle{\color{Purple}{2\times3}}={\color{blue}{6}}\end{cases}}</math>||3    | ||
| + | |- | ||
| + | |<span style="color:Purple">9</span>7344||7344 | ||
| + | |- | ||
| + | |<span style="color:Purple">3</span>    ||<span style="color:#0000FF">6</span>    | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Then, start from 9, because 9 is the fifth digit. | ||
| + | |style="text-align:right;"|אז תתחיל בט' כי הט' אות חמיש‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Say: what square is in 9? Know that the square in 9 is [generated from] 3, because 3 times 3 is 9 and you have nothing left. | ||
| + | |style="text-align:right;"|ואז תאמר כמה הריבוע בט' ודע שהריבוע בט' הוה ג' כי ג' פעמי' ג' זהו ט' ולא נשאר לך מאומה | ||
| + | |- | ||
| + | | | ||
| + | ::Write the 3 beneath the 9 and above the 9, like this: | ||
| + | |style="text-align:right;"|והג' תכתוב תחת הט' ועל הט' כזה | ||
| + | |- | ||
| + | | | ||
| + | :{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |3|| || || || | ||
| + | |- | ||
| + | |9||7||3||4||4 | ||
| + | |- | ||
| + | |3|| || || || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ג|| || || || | ||
| + | |- | ||
| + | |ט||ז||ג||ד||ד | ||
| + | |- | ||
| + | |ג|| || || || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Next, double the 3; the result is 6. | ||
| + | |style="text-align:right;"|ואז תכפול הג' לאחריה והוה ו‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Write it backwards beneath the 7 and shift the upper 3 above the 7 backwards, but do not double it as you do with the bottom 3. This is its diagram. We erase the 9, like this: | ||
| + | |style="text-align:right;"|ותשים לאחריה תחת הז' והג' שלמעלה תמשוך לאחריה על הז' אבל [לא] תכפול כמו שתעשה לג' תחתונ' והילך צורתו ונמחק הט' כזה | ||
| + | |- | ||
| + | | | ||
| + | :{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |3|| || || | ||
| + | |- | ||
| + | |7||3||4||4 | ||
| + | |- | ||
| + | |6|| || || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ג|| || || | ||
| + | |- | ||
| + | |ז||ג||ד||ד | ||
| + | |- | ||
| + | |ו|| || || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Say: how many times is 6 in 7? It is only one time and 1 remains. | ||
| + | |style="text-align:right;"|ואז תאמ' כמה פעמי' ו' בז' ואינ' אלא פעם א' ועוד נשאר א‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Write the 1 resulting from the 7 above the 3 before the 7 and beneath the 3. | ||
| + | |style="text-align:right;"|ואות' א' שעולה מן הז' תכתוב על הג' שלפני הז' ותחת הג‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Erase the 7 and write instead the 1 remaining from the 7. This is the diagram: | ||
| + | |style="text-align:right;"|ונמח' הז' ותכתו' במקומ' א' הנשארת מן הז' שלא מגיע נמי לז' וזה וצורת' כזה | ||
| + | |- | ||
| + | | | ||
| + | :{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |3||1|| || | ||
| + | |- | ||
| + | |1||3||4||4 | ||
| + | |- | ||
| + | |6||1|| || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ג||א|| || | ||
| + | |- | ||
| + | |א||ג||ד||ד | ||
| + | |- | ||
| + | |ו||א|| || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Then, take the 1 also once from 13; 12 still remains. | ||
| + | |style="text-align:right;"|ואז תקח הא' נמי א' פעם מן ג"א ועדיין הוה ב"א | ||
| + | |- | ||
| + | | | ||
| + | :{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |3||1|| || | ||
| + | |- | ||
| + | |1||2||4||4 | ||
| + | |- | ||
| + | |6||1|| || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ג||א|| || | ||
| + | |- | ||
| + | |א||ב||ד||ד | ||
| + | |- | ||
| + | |ו||א|| || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Next, double the 1 beneath the 2 [and shift it] back beneath the 4; it is 2. | ||
| + | |style="text-align:right;"|ואז תכפול הא' שתחת הב' לאחריה תחת הד' ויהא ב‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Shift the 6 also backwards, but do not double it, because you already doubled the 6. Write the 6 beneath the 2, where the 1 was written. | ||
| + | |style="text-align:right;"|והו' תשים ג"כ לאחרי' אבל לא תכפול שכבר כפלת הו' ותשים אות' ו' תחת הב' שכבר עומד שם הא‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Shift the 1 above the 2 backwards as well, above the 4, but do not double it; and the 3 instead of the 1 above the 2. Like this: | ||
| + | |style="text-align:right;"|והא' למעלה על הב' נמי תמשוך לאחריה על הד' ולא תכפול והג' במקו' הא' על הב' כזה | ||
| + | |- | ||
| + | | | ||
| + | :{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | | ||3||1|| | ||
| + | |- | ||
| + | |1||2||4||4 | ||
| + | |- | ||
| + | | ||6||2|| | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | | ||ג||א|| | ||
| + | |- | ||
| + | |א||ב||ד||ד | ||
| + | |- | ||
| + | | ||ו||ב|| | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Then, say: how many times is 6 in 12? It is twice in it. | ||
| + | |style="text-align:right;"|ואז תאמר כמה פעמים ו' בב"א פי' בי"ב והוה בו ב' פעמים | ||
| + | |- | ||
| + | | | ||
| + | ::Write the 2 above the first 4 and beneath the 4. | ||
| + | |style="text-align:right;"|אות' ב' תכתוב על הד' הראשונ' ותחת הד‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Erase the 12 and the bottom 6 as well. It is like this: | ||
| + | |style="text-align:right;"|ותמחוק הב"א ויהא צורתו כזה והו' שלמט' נמי תמחוק ויהא כזה | ||
| + | |- | ||
| + | | | ||
| + | :{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |3||1||2 | ||
| + | |- | ||
| + | | ||4||4 | ||
| + | |- | ||
| + | | ||2||2 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |ג||א||ב | ||
| + | |- | ||
| + | | ||ד||ד | ||
| + | |- | ||
| + | | ||ב||ב | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |- | ||
| + | |colspan="2" | | ||
| + | :{| | ||
| + | |- | ||
| + | |rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{Purple}{7-\left({\color{blue}{1}}\times6\right)=}}{\color{blue}{1}}}</math>||3<span style="color:#0000FF>1</span>  ||rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{Purple}{13-{\color{blue}{1}}^2=}}{\color{blue}{12}}}</math>||31  ||rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{Purple}{2\times1=}}{\color{blue}{2}}}</math>|| 31 ||rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{Purple}{12-\left({\color{blue}{2}}\times6\right)=0}}}</math>||31<span style="color:#0000FF>2</span> | ||
| + | |- | ||
| + | |<span style="color:#0000FF>1</span>344||<span style="color:#0000FF>12</span>44||<span style="color:Purple">12</span>44|| 44 | ||
| + | |- | ||
| + | |6<span style="color:#0000FF">1</span>  ||6<span style="color:Purple">1</span>  || 6<span style="color:#0000FF">2</span> || <span style="color:#0000FF">2</span>2 | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | ::Then, take the 2 also twice; the result is 4 and nothing remains. Take the other 2 twice as well from the 4; you have nothing left. | ||
| + | |style="text-align:right;"|ואז תקח הב' נמי ב' פעמים והוה ד' ולא נשאר מאומ' והב' השנייה נמי תקח ב' פעמים מן הד' ואז לא נשאר לך מאומא | ||
| + | |- | ||
| + | | | ||
| + | ::We find that the factor was 312 times 312 and the resulting total is 97344. | ||
| + | |style="text-align:right;"|נמצא הפרט הוה ב'א'ג' פעם ב'א'ג' ויוצא ממנ' כלל ד'ד'ג'ז'ט‫' | ||
| + | |- | ||
| + | |colspan="2" | | ||
| + | :{| | ||
| + | |- | ||
| + | |<math>\scriptstyle\xrightarrow{\scriptstyle\begin{cases}\scriptstyle{\color{Purple}{4-\left(2\times2\right)}}=4-4={\color{blue}{0}}\\\scriptstyle{\color{Purple}{4-2^2}}=4-4={\color{blue}{0}}\end{cases}}</math>||<span style="color:#0000FF>312×312=97344</span> | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ודוק ותובין לעולם בדרך זה | ||
| + | |- | ||
| + | |} | ||
| + | |||
| + | == Notes == | ||
| + | |||
| + | <div style="text-align: right;"><div class="mw-collapsible mw-collapsed"><div class="mw-collapsible-content"> | ||
| + | <br> | ||
| + | <references group=note/> | ||
| + | </div></div></div> | ||
| + | |||
| + | == Apparatus == | ||
| + | <div class="mw-collapsible mw-collapsed"><div class="mw-collapsible-content"> | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|<references /> | ||
| + | |- | ||
| + | | | ||
| + | |} | ||
| + | </div></div> | ||
| + | |||
| + | == Appendix I: Glossary of Terms == | ||
| + | <div class="mw-collapsible mw-collapsed"><div class="mw-collapsible-content"> | ||
| + | {| | ||
| + | |- | ||
| + | |to know | ||
| + | |style="text-align:right;"|לידע, דע כי, דע שתדע ש | ||
| + | |- | ||
| + | |to explain | ||
| + | |style="text-align:right;"|לפרש, ביארתי | ||
| + | |- | ||
| + | |chapter | ||
| + | |style="text-align:right;"|שערים | ||
| + | |- | ||
| + | |teaching | ||
| + | |style="text-align:right;"|הקבלות | ||
| + | |- | ||
| + | |one who calculates | ||
| + | |style="text-align:right;"|המחשב, המפתח | ||
| + | |- | ||
| + | |calculation | ||
| + | |style="text-align:right;"|החשבונות | ||
| + | |- | ||
| + | |numeral | ||
| + | |style="text-align:right;"|חשבון, אותיות, ציפרא | ||
| + | |- | ||
| + | |zero | ||
| + | |style="text-align:right;"|גלגל | ||
| + | |- | ||
| + | |place holder | ||
| + | |style="text-align:right;"|שומר המעלות | ||
| + | |- | ||
| + | |first rank | ||
| + | |style="text-align:right;"|מעלה הראשונה | ||
| + | |- | ||
| + | |units | ||
| + | |style="text-align:right;"|מעלת היחידים | ||
| + | |- | ||
| + | |third rank | ||
| + | |style="text-align:right;"|מעלה השלישית | ||
| + | |- | ||
| + | |hundreds | ||
| + | |style="text-align:right;"|מעלת המאות | ||
| + | |- | ||
| + | |fourth rank | ||
| + | |style="text-align:right;"|מעלה הרביעית | ||
| + | |- | ||
| + | |thousands | ||
| + | |style="text-align:right;"|מעלת האלפים | ||
| + | |- | ||
| + | |fifth rank | ||
| + | |style="text-align:right;"|מעלה החמישית | ||
| + | |- | ||
| + | |tens of thousands | ||
| + | |style="text-align:right;"|מעלת הרבבות, י' אלפים | ||
| + | |- | ||
| + | |sixth rank | ||
| + | |style="text-align:right;"|מעלה ששית | ||
| + | |- | ||
| + | |hundreds of thousands | ||
| + | |style="text-align:right;"|מעלת מאה אלפים | ||
| + | |- | ||
| + | |seventh rank | ||
| + | |style="text-align:right;"|מעלה שביעית | ||
| + | |- | ||
| + | |millions | ||
| + | |style="text-align:right;"|מעלת אלף אלפים | ||
| + | |- | ||
| + | |smaller than | ||
| + | |style="text-align:right;"|קטן מן | ||
| + | |- | ||
| + | |greater than | ||
| + | |style="text-align:right;"|גדול מן | ||
| + | |- | ||
| + | |more than | ||
| + | |style="text-align:right;"|יותר מ | ||
| + | |- | ||
| + | |to exceed by | ||
| + | |style="text-align:right;"|יותר על, יתיר על, עודף על, למעלה מ | ||
| + | |- | ||
| + | |bottom numeral | ||
| + | |style="text-align:right;"|התחתונה, תחתונה | ||
| + | |- | ||
| + | |upper numeral | ||
| + | |style="text-align:right;"|העליונה, עליונה | ||
| + | |- | ||
| + | |infinitely, endlessly | ||
| + | |style="text-align:right;"|עד אין מספר | ||
| + | |- | ||
| + | |times | ||
| + | |style="text-align:right;"|פעמים | ||
| + | |- | ||
| + | |to multiply | ||
| + | |style="text-align:right;"|לחשוב, לכפול זע"ז | ||
| + | |- | ||
| + | |rank | ||
| + | |style="text-align:right;"|מעלות, מעלה | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|המעלה שנייה | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|עליונה | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|שיט' | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|שבשיט' עליונ', שיטות עליונות, שיטות העליונות | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|שיט' ראשונ' | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|השיטה התחתונה | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|דומיון, דמיון | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|מגיע ל | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|עולה, יעלה, יעלה בידך, מה שיעלה בידך, העולה בידך | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|יצא | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|נקראת, נקרא | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|יקראו | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|אות, האות | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|מניינם, מניין | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|תחשוב | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|חשוב | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|חשוב אותו | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|חשבונך | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|תאמר, אמור | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ישאלך | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|שואל | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|תשיב אל לבך | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|לכתוב, כתוב, כתוב (אותו / אותה / ב), כתבתי, כתבת, תכתוב (אותו), נכתוב, אכתוב לך, כותבין | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|כתיבה | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|שכתבת | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|רשום, ארשום לך | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|חרו[ת]ים | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|העתקתי | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|לראות | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|שאראך | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|אדירין | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|חברם יחד | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|לחבר, לחבר ... עם, תחבר...אל, תחבר... על, תחבר... ה, חבור... על, חבור אל ה, נחבר... עם, נחבר... על, אחבר | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|לחבר כמה חשבונות יחד | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|חבורם, חבורו, חיבור שחברנו | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|הטור מן החיבור | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|צירופו | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|תצרף, צרוף (...יחד), צורפם יחד, צרפם (יחד) | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|נוסיף... על, נוסיף... עליה | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|מוסיף ...על, מוסיף... עליה | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|בשער החיבור, שער חיבור | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|המחובר | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|השליך, השלך (...מ / ה), השלך מידך, קח ממנו, תקח ... מן, קח מה ש | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|השלך ט"ט... מ, השלך ... בט"ט | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|נשאר, ישאר, ישאר בידך, ישאר לך, הנשארת | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|בלי חסרון ויתרון | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ומה שנשאר לך, ומה שנשמר לך | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|הנותרת לי | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|משקלו, משקל ה, משקלים | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|אבני המשקל, אבן הראשון | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|תכפול יחד, לכפול יחד | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|תכפול | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|כפל | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|כפל קטן | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|מה שבא מן הכפל | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|בשער כפל | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|לוח | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|חצי לוח | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|מתוקנת | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|שער הכפל קטן, שער כפל קטן | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ציור | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|צורתו | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|עברת על ה, תעבור | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|תקח ה, קח ה, קח בידך, קח בידך ל, יקח ה... מה | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ומהם תבין | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|מרחק מן, המרחק עד, מרחקים | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|מכל אחד, מן כל אחד ואחד | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|זה על זה | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|דהיינו, היינו | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|הילך | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|היאך | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|המניין הקטון, מניין קטון, המנין הקטן, האות הקטן | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|אות הגדול | ||
| + | |- | ||
| + | |tens | ||
| + | |style="text-align:right;"|העשיריות | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ספר | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|גלחות, בגלחת | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|מחוק | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|עניין | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|כלל | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|פי' | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|המותר מ, עודף עליו | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|לעשות, עשה, תעשה, עשיתי | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|כאשר, כש | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|עתה | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|תדיר | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|לעולם | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ולפעמים | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|לא ... כלל | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|לא ימוש מפיך | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|תוכל ל | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|כל כמה שתוכל | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|אינך צריך | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|צריך ל | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|צריך ש | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|תלוה לו, הלוית לו | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|תרצה (ל), תחפוץ ל, בקשת ל, רצונך, בקשנו ל | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|אשר בקשת, מבוקש | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|מתכונך | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|מכוון | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|כוינת חשבונך | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|אשכילך | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|תשכיל, תחכם | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|מושכל ראשון | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|עיין | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|תקיש | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ודוק | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|להודיעך ש | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|למצוא, תמצא (ה), נמצא, מצאתי, נמצא ה | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|לזכרון | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|וככלות | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|תשלם | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|המלאכה | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|סליק | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|הוי אומר | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|בדרך הזה, דרך אחרת ל | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ואז, אז | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|כבר | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|כדי ש | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|מה ש | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|כל מה ש, כל אשר | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|אשר ב | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|אף כי | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|כי | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|לכך | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|והנה | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|נמי | ||
|- | |- | ||
| | | | ||
| − | + | |style="text-align:right;"|אחר כך, אח"כ | |
| − | |||
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | |style="text-align:right;"|ואם... אז | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | |style="text-align:right;"|אם | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | |style="text-align:right;"|אפילו | |
| − | |||
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | |style="text-align:right;"|רק | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | |style="text-align:right;"|כמה | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | |style="text-align:right;"|יהיה בכל פעם | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | |style="text-align:right;"|פעמים | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | |style="text-align:right;"|לכל היותר | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | |style="text-align:right;"|יותר מן | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | |style="text-align:right;"|מותר ה... מ | |
| − | |style="text-align:right;"| | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | + | |style="text-align:right;"|פחות מ, פחותים מ, מה שלמטה מן, למטה מ | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | |style="text-align:right;"|הרבה כל כך | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | |style="text-align:right;"|ויהיה, ויהי, ויהא | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | |style="text-align:right;"|שם | |
| − | |||
| − | |style="text-align:right;"| | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | + | |style="text-align:right;"|יש | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | |style="text-align:right;"|הרי | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | |style="text-align:right;"|ויהיה הכל | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | |style="text-align:right;"|הוה | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | |style="text-align:right;"|שהם | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | |style="text-align:right;"|אני | |
| − | |||
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | |style="text-align:right;"|הוא, היא | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | |style="text-align:right;"|או כלך לדרך זו, או כלך בדרך זו | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | |style="text-align:right;"|זה, זה ה | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | |style="text-align:right;"|כזה | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | |style="text-align:right;"|הזה | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | |style="text-align:right;"|אילו ה | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | |style="text-align:right;"|האחרונים | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | |style="text-align:right;"|חשבונות, חשבון | |
| − | |||
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | |style="text-align:right;"|שבידך | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | |style="text-align:right;"|בא לידך מ | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | |style="text-align:right;"|לפניך, מה שלפניך | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|הסמוכ' לה, הסמוך ל |
|- | |- | ||
| | | | ||
| − | + | |style="text-align:right;"|מצד אותיות של שמאל | |
| − | |||
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | |style="text-align:right;"|לצד שמאל, בצד שמאל (ל), לשמאל | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | |style="text-align:right;"|אצל, אל | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | |style="text-align:right;"|נגד | |
| − | |||
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | |style="text-align:right;"|במקום | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | |style="text-align:right;"|אחר | |
| − | |||
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | |style="text-align:right;"|עוד | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ועוד |
|- | |- | ||
| | | | ||
| − | + | |style="text-align:right;"|וכן, ג”כ | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | |style="text-align:right;"|וכפי זה | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | |style="text-align:right;"|כגון | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | |style="text-align:right;"|כאילו | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | |style="text-align:right;"|כמו כן | |
| − | |||
| − | |||
| − | |||
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | |style="text-align:right;"|כל כיוצא בזה | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | |style="text-align:right;"|שלאחריו, לאחריה, שלאחריה | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | |style="text-align:right;"|שלפניה, לפני ה, קודם ה | |
| − | |||
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | |style="text-align:right;"|מן ה | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | |style="text-align:right;"|תחת, תחת ה | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | |style="text-align:right;"|למטה, שלמטה | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | |style="text-align:right;"|למעלה, למעלה מזו | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | |style="text-align:right;"|לעיל | |
| − | |||
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | |style="text-align:right;"|שאין בו ממש | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | |style="text-align:right;"|בקלות | |
| − | |||
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | |style="text-align:right;"|ולא ... אלא | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | | | + | |style="text-align:right;"|בלא שום |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|זכר ל |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|דבר |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כל דבר |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|את"ל |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|בע"ה |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|לישב על אופניו |
| − | |||
|} | |} | ||
| − | + | </div></div> | |
| − | + | ||
| − | + | == Appendix II: Bibliography == | |
| + | '''''Anonymous'''''<br> | ||
| + | '''''Sefer Ṣifra'''''<br> | ||
| + | |||
| + | |||
| + | '''Manuscripts:''' | ||
| + | :1) Budapest, Magyar Tudományos Akadámia, Ms. Kaufmann A 520/10 (IMHM: f 15170), ff. 179-200; 217-218 (16th century) | ||
| + | :[https://www.nli.org.il/he/discover/manuscripts/hebrew-manuscripts/viewerpage?vid=MANUSCRIPTS&docid=PNX_MANUSCRIPTS990001919280205171-1#$FL53195101 Kaufmann A 520/10] | ||
| + | :2) Cincinnati, Hebrew Union College 890/1 (IMHM: f 30901), ff. 4r-18v (Hanau, 1641)<br> | ||
| + | :[https://www.nli.org.il/he/discover/manuscripts/hebrew-manuscripts/viewerpage?vid=MANUSCRIPTS&docid=PNX_MANUSCRIPTS990001271690205171-1#$FL26622640 Cincinnati 890] | ||
| + | :3) Mantova, Comunità Ebraica MS ebr. 8/16 (IMHM: f 788), ff. 69r-71v (Porto, 1464-1471) | ||
| + | :[http://digilib.bibliotecateresiana.it/sfoglia_ebraici.php?g=CME_001_030&sg=CME008&identifier=MN0035-EBRA-cme008_2 Mantova 8] | ||
| + | :4) Moscow, Russian State Library, Ms. Guenzburg 714/4 (IMHM: f 27994), ff. 64r-83v (17th-18th century) | ||
| + | :[https://www.nli.org.il/he/discover/manuscripts/hebrew-manuscripts/viewerpage?vid=MANUSCRIPTS&docid=PNX_MANUSCRIPTS990000876620205171-1#$FL79171989 Guenzburg 714] | ||
| + | :5) München, Bayerische Staatsbibliothek, Cod. hebr. 394/4 (IMHM: f 1223), ff. 109v-127r (1566) | ||
| + | :[http://daten.digitale-sammlungen.de/~db/0002/bsb00021775/images/index.html?id=00021775&groesser=&fip=eayayztsxdsydeayaeayaxseayaenxdsydxdsydqrs&no=11&seite=239 München Cod. hebr. 394] | ||
| + | :6) New York, Jewish Theological Seminary Ms. 2634/6 (IMHM: f 28887), ff. 94r-124v<br> | ||
| + | :[https://www.nli.org.il/he/discover/manuscripts/hebrew-manuscripts/viewerpage?vid=MANUSCRIPTS&docid=PNX_MANUSCRIPTS990001053920205171-1#$FL30386852 JTS 2634] | ||
| + | :7) Oxford, Bodleian Library MS Mich. 60/11 (IMHM: f 22085), ff. 151r-176v (cat. Neub. 2171); (Frankfurt am Main, 1537) | ||
| + | :8) Oxford, Bodleian Library MS Mich. 440/4 (IMHM: f 19984), ff. 114v-124v (cat. Neub. 2170); (1645) | ||
| + | :9) Paris, Bibliothèque Nationale heb. 1088/1 (IMHM: f 14921), ff. 1r-23v (15th century)<br> | ||
| + | :[http://gallica.bnf.fr/ark:/12148/btv1b10545031p/f8.image heb.1088] | ||
| + | :10) Paris, Séminaire Israélite de France (École Rabbinique) 158/1 (IMHM: f 4102), ff. 197r-211v (1604) | ||
| + | :[https://www.nli.org.il/he/discover/manuscripts/hebrew-manuscripts/viewerpage?vid=MANUSCRIPTS&docid=PNX_MANUSCRIPTS990001831980205171-1#$FL62298918 École Rabbinique 158] | ||
| + | :11) Warszaw, Żydowski Instytut Historyczny 288/1 (IMHM: f 12013), ff. 1r-5v (15th century) | ||
| + | :12) Warszaw, Żydowski Instytut Historyczny 295/4 (IMHM: f 10129), f. 56v (16th century) | ||
| + | |||
| + | <span style=color:blue>The transcript is based mainly on manuscript München 394</span> | ||
| − | + | '''Bibliography:'''<br> | |
| − | + | *Steinschneider, Moritz (Moshe). 1893–1901. Die Mathematik bei den Juden. Berlin-Leipzig-Frankfurt: Kaufmann; repr. Hildesheim: G. Olms, 1964 and 2001. pp. 216-217 [i478-i479] | |
| − | + | *———. 1905. Mathematik bei den Juden, Band II: 1551-1840. Monatsschrift für die Geschichte und Wissenschaft des Judenthums 49, pp. 85-86. repr.: ed. Gad Freudenthal, Hildesheim, Zürich, New York: Olms, 2014, pp. 8-9. | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
Latest revision as of 06:58, 5 July 2024
Contents
- 1 Prologue
- 2 Introduction
- 3 Chapter One: Multiplication of Units - Shortcuts
- 4 Chapter Two: Addition
- 5 Chapter Three: Subtraction
- 6 Chapter Four: Multiplication
- 7 Chapter [Five]: Multiplication in a Table [Gelosia]
- 8 Chapter Six: Division
- 9 Chapter Seven: Proportions
- 9.1 Word Problems
- 9.1.1 Pricing Problems - Find the Price
- 9.1.2 Boiling Problem
- 9.1.3 Find a Quantity Problems - Whole from Parts
- 9.1.4 Joint Purchase Problems - If You Give Me
- 9.1.5 Find a Quantity Problems - Whole from Parts
- 9.1.6 Shared Work Problems - Filling/Draining a Vessel
- 9.1.7 Multiple Quantities Problems - Selling Eggs
- 9.1.8 Proportional Division - Inheritance
- 9.1.9 Payment Problem - Digging a Hole
- 9.1.10 Find a Quantity Problem - Stolen Purse
- 9.1.11 Find a Quantity Problem - How Much Problem - Amount of Money
- 9.1.12 Divide a Quantity - Loans Repayment
- 9.1.13 Multiple Quantities - Weights
- 9.1.14 Divide a Quantity - Money
- 9.1.15 Find a Quantity Problem - Whole from Parts - Fish
- 9.1.16 Multiple Quantities - Selling Cloth
- 9.1.17 Partnership Problems - for the Same Time - Three Partners
- 9.1.18 Multiple Quantities
- 9.1.19 Motion Problem - Pursuit
- 9.1.20 Ordering Problem - Stock of Coins
- 9.1.21 Proportional Division - Three Men Sharing Food
- 9.1.22 Guessing Problem - Chosen Number
- 9.1.23 Multiple Quantities - Men, Women, Children
- 9.1.24 Double False Position Find a Quantity Problem - How Much Problem - Money
- 9.2 Additional Word Problems
- 9.1 Word Problems
- 10 Chapter On Cubic Roots [P1088 2r-v]
- 11 Here is a Chapter, Called the Chapter on Square Roots [P1088 6v-7v]
- 12 Notes
- 13 Apparatus
- 14 Appendix I: Glossary of Terms
- 15 Appendix II: Bibliography
Prologue |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| [MS Paris, Séminaire Israélite de France (École Rabbinique) 158/1 (IMHM 4102) f. 197r, lines 1-16] | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| This is a book on mathematics [called] Ṭeshifra. | זה ספר חכמת התשבורת [הנקרא] טשיפרא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Its cause: I myself, already from youth, my soul desired to acquire wisdom and to seek for arithmetic, if only I will find them in a book engraved by a pen of a skilled writer. For the reason that this is an external science, which is not found before us in Israel and the very least that is available is gathered inside the house in utmost discretion, my thoughts therefore aroused me to collect the sayings from any one that has them […] for recollection. | סיבתו אני את לבי גם מנעורי גרסה נפשי לדעת חכמה ובקש חשבון ומי יתן ואמצאם בספר ויחקו בעט סופר מהיר [note 1] ולסבה שזאת היא חכמת החיצוניות לא נמצאת לפנינו בישראל והמיעוט שבמיעוט הנמצא נאסף אל תוך הבית בחדרי חדרים לכן העירוני רעיוני ללקט האמרים מיד כל אשר ימצא אתו ולכל[...] לזכרון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Before I start, I ask all its readers not to condemn me and say: what is it with you? Go study havayot d'Abbaye ve-Rabba! God forbid […]. Since I did not undertake it for any cause or purpose, but sharpening the mind and obtaining arithmetic by accident and learning and by the wittiness of Abbaye ve-Rabba primarily. On that said those who speak in parables: come to Ḥeshbon [Numbers 21, 27]. As is written in Maimonides, chapter five of the introduction to Tractate Avot: | וקודם שאתחיל אבקש מיד כל רואיו לבל יכרעני לכף חובה ולומר מה לך פה כלך בהוייות דאביי ורבא חלילה וחס [לי'] לזרעא דאבא כי לא לקחתי זאת לשום עיקר ותכלית רק לחדד השכל ולבא בחשבון במקרה ושנייה ובחידוד דאביי ורבא בעצם וראשונה ועל זאת אמרו המושלים באו חשבון [note 2] כמו שכתוב הרמבם ז"ל פרק חמישי מה[קדמת] ה פרקי אבות [note 3] וז"ל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| What has no benefit in it for that purpose – as the arithmetic problems, the Book of Conics, the numerous techniques for questions on geometry, the measuring of weights, and many like those – the intention in them is to sharpen the mind and train the rational faculty through methods of proof, until a person acquires the knowledge of demonstrative reasoning from other, and this will be his way whereby he will reach the knowledge of the truth of His existence, The Exalted. | ומה שאין בו תועלת לו בתכלית ההוא כשאלות החשבון וספר החר[וט]ים והתחבולות הרבות משאלות אל ההנד[ס]ה ומשיכת המשקלים והרבה כיוצא בא[ילו] יהיה הכוונה בהם לחדד השכל ולהרגיל כח השכלי בדרכי המופת עד שיגיע לו לאדם קניין ידיעת ההיקש המופתי מזולתו ויהיה לו זה הדרך שיגיע בה לידעת אמיתות מציאתו ית' עכל' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| So, for my part it will not be inaccessible. | גם מצדי לא יבצר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Introduction |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| With good luck | [1]בטוב גדא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| I shall start this | אתחיל דא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Sefer Ṣifra | ספר ציפרא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| To know and explain the book of number that has 9 chapters. | לידע ולמפרש ספר המספר שיש בו ט' שערים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| First I shall explain the teachings that the one who calculates should know, so that he can know all the calculations [practice]. | ואפרש תחלה הקבלות אשר צריך לידע המחשב הוא המפתח ויכול אח"כ לידע כל החשבונו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
The Positional Decimal System |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The numerals | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Know that you do not have any number [= numeral] smaller than one and you do not have any number [= numeral] greater than nine. | נידע כי אין לך חשבון קטן מן א' שהוא ואין לך חשבון שהוא גדול מן ט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| By these nine numerals from א to ט [the nine first Hebrew letters] all numbers in the world [are formed] infinitely. How?: | ובאילו ט' אותיו' מא' ועד ט' יש בו כל החשבונות שבעולם עד אין מספר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The written ranks [= decimal places] | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כיצד מעלה ראשונה היא נקראת מעלת היחידים כי כאשר יקראו האות כך מניינם פי' א' אחד ב' שנים וכן עד ט' שהיא תשע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכשתחשוב עשרה אז צריך אתה לכתוב ב' מעלות א' במעלה שנייה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולפניו ציפרא שהוא גלגל כזה 0 שאין בו ממש אלא שומר המעלות וזכר לדב' כגלגל לפני רוח [note 4] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
0א הוא י' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אא הוא י"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כי הא' הראשונה מן היחידים שהיא במעלה ראשונה וא' שנייה היא מן העשיריו' הרי י"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
בא הרי י"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
גא הרי י"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וככה עד טא שהוא י"ט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
0ב הוא עשרים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אב אחד ועשרים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
בב שנים ועשרים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וככה עד תשע ועשרי' שהוא טב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ושלישי הוא מעלות המאות כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
00א שהוא ק' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
00ב הוא ר' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
00ג הוא ש' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וככה עד ט' מאות שהוא 00ט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אאא הוא קי"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
א0א הוא ק"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומעלה הרביעי' היא מעלת האלפי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
000א הוא אלף | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
000ב הוא ב' אלפי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וככה עד ט' אלפים שהוא 000"ט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומעלה החמישית היא מעלת הרבבות שהוא י' אלפי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וששה מעלה הוא מאה אלפי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ושביעי' אלף אלפים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| And so you can write endlessly. | וככה עד אין מספר תוכל לכתוב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Every rank is ten times the preceding rank. | וכל מעלה היא עשרה פעמי' יותר מן מעלה שלפניה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם ישאלך השואל 0ב0ג כמה הן | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תשיב אל לבך כי הגלגל אין לו מניין רק שומר המעלות והנה החשבון עשרים וג' אלפים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Thus, I have explained to you the number and the meaning of the writing. | והנה ביארתי לך מניין וענין הכתובי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 1 2 3 4 5 6 7 8 9 0 10 20 30 |
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Chapter One: Multiplication of Units - Shortcuts |
שער הכפל הקטן | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If you wish to know how much is the result of 9 times 8, or 5 times 9, or 6 times 9 and so on for all that is less than ten, I will teach you: | ואם תחפוץ לידע כמה עולה ט' פעמים ח' או ה'פ'ז' או ו' פעמי' ט' [וכן כל כיוצא בזה שכל אחד פחות מעשרה][2] אשכילך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם תחפוץ לידע כמה ט’ פעמי' ט’ | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אז כתוב כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואח"כ חשוב כמה יותר על עשרה ח' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואת' ח' כתוב אצל הט' העליונה [3]בצד שמאלית כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואח"כ חשוב כמה ט' העליונה יותר על ח' ותמצא רק אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
[אותו א’ כתוב בצד ט’ התחתונה][4] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכמה ט' התחתונה יותר על הח' ותמצא רק אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
[כתוב אותה א’ ג”כ בצד ט’ תחתונה כזה][5] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותחשוב א'פ'א' היינו אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כתוב א' לפני הח' כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
[ומחוק הט' למטה ולמעלה][6] ויהיה אחד ושמונים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן אם תרצה לידע כמה ט' פעמים ח' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כתוב כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וחשוב כמה ח'ט' יותר על עשרה דהיינו ז' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כתוב הז' אצל הט' כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וחשוב כמה ט' עודף על ז' דהיינו ב' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכמה הח' עודף על ז' א' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
[וכתוב כזה][7] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תכפול א' על ב' היינו ב' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אות' ב' כתוב קודם הז' כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נמצא ט' פעמי' ח' יהיה ע"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
[8]דומיון אם תחפוץ לידע כמה ו' פעם ו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אז כתו' הו' תחת הו' כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואז תאמר כמה השני ו' למעלה מי' זהו ב' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואותו ב' תכתו' לאחר הו' כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואז תאמ' כמה הו' יתיר על הב' זהו ד' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והו' השנייה נמי למעל' מב' זהו ד' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואז תכפול ד' פעמי' ד' זהו ו"א פי' י"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואז תכתוב שים הא' על הב' כי הב' מעלה שניי' ויהיה ג' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועוד הוה ו' ואות' ו' תכתו' לפני הג' כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נמצא שו' פעמ' ו' עולה ו"ג פי' ל"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Always do as this. | וכן עשה לעולם[9] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Multiply always the excess of this by the excess of this and write the result before the excess [of the sum of the multipliers over ten], then you will become wise in all that you do. | וכן לעולם תכפול המותר מזה ומזה והעולה כתו' קודם העודף עליו ואז תשכיל בכל אשר תעשה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Another example that I translated from a Latin book | [10]דמיון אחר שהעתקתי מספר גלחות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Sum together the two numerals, subtract a ten, then write the small number. | חברם יחד הב' אותיות והשליך עשרה והמניין הקטון כתוב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Multiply together the differences of each from ten and write [the product] before the small number that you have. | ואח"כ תכפול יחד החשבון שיש מכל אחד עד עשרה וכתוב זה לפני המנין הקטן שבידך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If this product is of two ranks, add the second rank to the number that you have. | ואם בא לידך מהכפל הזה ב' מעלות אז תחבר המעלה שנייה אל המניין שבידך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| As these calculations that are written in front of you, from which you will become wiser: | כגון אילו החשבונות החרו[ת]ים לפניך ממנו תשכיל ותחכם עוד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Or in this way: write zero 0 before the smaller of the two numerals. | או כלך לדרך זו כתוב לפני המניין קטון שבשני אותיות גלגל כזה 0 | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כגון את"ל ז'פ'ח' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כתוב כזה 0ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וקח ממנו מה שבא מן הכפל שתכפול המניין מאות הגדול עד עשרה עם האות הקטן | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כגון מה שלפניך אמור ז'פ'ב' הם ד"א ותקח ד"א מן 0"ז ונשאר ו"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכגון אילו[11] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If you want to know and calculate how much is 3 times 3, or 4 times 4, et cetera, which even their sum does not reach ten, I will teach you how to do so: | ואם תרצה לחשוב כמה ג' פעמי' ג' ד'פ'ד' וכן כל כיוצא בזה שאפילו צירופו אינו מגיע לעשרה אשכילך לעשות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Lend to it as much as needed so that it will be one more than ten. | שתלוה לו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| When your calculation is complete, subtract what you have lent to it and the required remains. | וככלות חשבונך קח [מה שהלוית לו][12] וישאר מתכונ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון ד'פ'ד' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה תלוה לו ג' שיעלה י"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והשלך עשרה וישאר בידך א' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ורשום כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואח"כ חשוב כמה ד' הראשו' יותר על הא' הוי אומר ג' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן ד' השנייה ג"כ יותר ג' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפול ג' על ג' הרי ט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כתוב הט' לפני הא' כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה קח ג' שהלוית וישאר ו'א' [והם י"ו][13] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותמצא חשבונך מכוון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Another property: if you want to know how much is 3 times 3, or 4 times 4, et cetera, which even their sum does not reach ten, I will teach: | עניין אחר אם תרצה לידע כמה ג"פ"ג או ד"פ"ד וכן כל כיוצא בזה שאפי' צירופו אינו מגיע לעשרה אשכילך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דומיון אם תרצה לחשוב ד'פ'ד’ | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אז תכתוב אותו כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וחשוב כמה ד' ראשונה פחות מי' ויהיה ו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וחשוב גם השנייה כמה השנייה פחות מי' ותמצא ג"כ ו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואז תכפול ו' על ו' ויעלה בידך ו'ג' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אז חשוב כמה השני ד' פחותי' מעשר' ויהיה ב' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
השלך ב' ממעלה שנייה מהג' וישאר לך ו'א' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נמצא ד'פ'ד' הוא ו'א' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן כולם וא"ק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If the numbers that you want to multiply one by the other are less than 10, when you sum them together: | [14]ואם יהיה המספר אשר תרצה לכפול זע"ז פחות מי' כשתצרף שני המספרים יחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דימיון אם בקשת לכפול ד' על ג' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אז תקח המרחק מן י' מכל אחד דהיינו מד' עד י' ו' ומג' עד י' ז' ופ"ז ב"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אותו ב"ד כתו' לזכרון [בד] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואח"כ צרוף ד' ג' יחד ויהיה ז' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אות ז' כתוב לצד שמאל אצל ב"ד כזה [בדז] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואח"כ השלך י' מצד אותיות של שמאל וישאר ב'א' היינו החשבון אשר בקשת לידע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכפי זה תקיש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה כתבתי זה אף כי הוא מושכל ראשון החשבון אשר בקשת להודיעך שאין בדרך הזה [...][15] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| I have found another method for the multiplication of units in another book: | [16]דרך אחרת לכפל קטן בספר אחר מצאתי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Or in this way: take the difference from 10 of each [of the units] that you want to multiply, multiply the two differences by each other and write the result for remembrance. | או כלך בדרך זו קח המרחק עד י’ מן כל אחד ואחד שתרצה לכפול יחד וחשוב אותו ב’ מרחקים זה על זה ומה שיעלה בידך כתוב לזכרון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Then, sum [the multipliers] together according to the chapter of addition, subtract 10 from the result, and write [the remainder] below to the left of what you have already wrote. | אחר כך צורפם יחד בשער החיבור והעולה בידך קח מה שלמטה מן י’ וי’ השלך מידך וכתוב אותו למטה בצד שמאל למה שכתבת כבר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Your calculation will be correct. | ויהיה חשבונך מכוון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון הנה רצונך לכפול ז’ פעמים ט’ | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
קח המרחק מן הז' עד י' דהיינו ג' והמרחק מן ט’ עד י’ דהיינו א’ ואמור ג’ פעמים א’ היינו ג’ | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אותו ג’ כתוב לזכרון כזה ג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אחר כך חבור ז' על ט' ויעלה ו"א פי' י"ו השלך הי’ והו’ קח בידך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכתוב אותה בצד שמאל אצל הג’ הנשארת ויהיה כזה גו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועיין | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון אחר הנה רצונך לכפול ו’ פעמים ח’ | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
קח המרחק מן ו’ עד י’ דהיינו ד’ והמרחק מן ח’ היינו ב’ ואמור ב’ פעמים ד’ היינו ח’ | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אותו ח' כתוב לזכרון [ח] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אחר כך חבור ו' על ח' ויעלה י”ד השלך י' וקח ד' בידך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכתוב ד' בצד שמאל אל ח' אשר כתבת כבר ויעלה מ”ח היינו חשבון ח'פ'ו' [חד] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עיין ודוק[17] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
[18]דמיון אחר אם תרצה לידע הפ"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
קח המרחק מן ה' עד י' ויהיה ה' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
צרפם יחד הה’ והו’ ויהיה א' השלך עשרה ונשאר לך א' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
צרפם 0"ב ויהיה ג' נמצא ל' ודוק[19] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Multiplication Table | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| I will write to you a precise table of multiplication of units, in order that you will be able to observe easily all that you want without any calculation, from 1 times 1 to 9 times 9. Note well. | והנה ארשום לך לוח מתוקנת על שער הכפל קטן כדי שתוכל לראות בקלות כל מה שתרצה בלא שום חשבון מן א' פעמי' א' עד ט' פעמים ט' ודו"ק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| I made a half table in order to find every number, since you do not need that much. | והחצי לוח עשיתי למצוא כל חשבון ואינך צריך הרבה כל כך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The chapter on the multiplication of units is completed. | סליק שער כפל קטן | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| You shall become wise in all that you do. | ואז תשכיל כל אשר תעשה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Chapter Two: Addition |
[20]שער החיבור | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם תרצה לחבר שנים ושבעים וג' מאות וג' אלפים עם שנים ותשעים וג' מאות וג' אלפי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תכתוב כך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תחבר ב' התחתונה על ב' העליונה ויהיה ד' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תחבר ט' התחתונה עם הז' ויהיה ו"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הו' תכתוב במעלה השנייה אחר הד' [שכתבת כזה][21] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והא' [הנשארת][22] תחבר לג'ג' הראשונה שהם זו למעלה מזו כזה במעלה השלישית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ויהיה הכל ז' במעלה השלישי' כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואח"כ תחבר הג'ג' האחרוני' [במעלה הרביעי'][23] [ויהיה חבורם][24] ו' [אות' ו' כתוב במעלה הרביעי' אחר דוז שכתבת][25] ויהיה כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
[ותשלם המלאכה ויהיה חבורו ארבע וששי' ז' מאות ו' אלפי' דהיינו כזה][26] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The highest rank of the sum exceeds the addend by one rank to the left at most, sometimes it does not exceed [its ranks] at all. | לעולם לא תמצא הטור מן החיבור אשר בעליונה עוברת על המחובר רק מעלה לשמאל לכל היותר ולפעמים לא תעבור כלל ול"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Here follows a chapter on addition, to add every thing and to establish its methods | [27]הילך שער חיבור לחבר כל דבר [..] ולישב על אופניו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Such as, if you wish to sum some numbers together. | כגון אם תרצה לחבר כמה חשבונות יחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Now, I sum one number and according to this you shall apply for others: | ועתה אחבר חשבון אחד ומהם תבין אחר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Write numeral by numeral [lit. letter by letter], but not as is written in the multiplication chapter, but write as I will demonstrate, with God's help. | ותכתו' אות תחת אות ולא תכתו' כאשר כותבין בשער כפל אלא תכתו' כזה שאראך בע"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דומיון בקשנו לחבר ע"ב וג' מאות וג' אלפי' עם צ"ב וח' מאות וט' אלפים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הילך היאך תכתוב אותו וצורתו כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נחבר ב' תחתונ' עם ב' עליונ' הרי ד' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אות' ד' נכתוב נגד ב' תחתונ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נחבר כמו כן ט' תחתונ' עם ז' עליונ' ויעלה ו"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נכתוב ו' תחת הט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונוסיף א' על אות שבשיט' עליונ' הסמוכ' לה ושם יש ג' ונוסיף א' עליה ויהיה כאילו נכתו' ד' במקום ג' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונחבר ד' עליונ' עם ח' תחתונ' ויעלה ב"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נכתו' ב' למטה תחת הח' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונוסיף א' על אות הסמוך לד' עליונ' ושם יש ג' ונוסיף א' עליה ויהא ד' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נחבר ד' עליונ' על ט' שלמטה ויעלה ג"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נכתוב ג' למט' תחת הט' וא' נכתוב לאחריה ויהיה כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Do not forget this: when the 1 remains from the first rank, I add it to the rank after it. Since the 1 I have left is also as tenths of the first rank, so I add it to it frequently. | זה הכלל לא ימוש מפיך כשמותר הא' משיט' ראשונ' אני מוסיף אות' על שיט' שלאחריה כי הא' הנותרת לי היא כמו כן מן העשיריות נגד שיט' ראשונ' לכך אני מוסיף אות' תדיר עליה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נמצא החיבור שחברנו לעיל הוא עולה ס"ד ומאתים וי"ג אלפים ודוק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| This diagram is from Latin and is called "addirin" [= addition]. | [28]ציור זה בגלחת ונקרא אדירין | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Checking Methods |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Now, know that I shall write you two checking method [lit. scales]: | [29]ועתה תדע שאכתוב לך שני משקלים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הא' השלך ט"ט כל כמה שתוכל מב' שיטות עליונות ומה שנשמר לך למטה מט' קח בידך לאבני המשקל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואח"כ השלך ג"כ השיטה התחתונה בט"ט ומה שנשאר לך אם משקלו כאבן הראשון דע שכוינת חשבונך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
משקל השני יקח השני שיטות העליונות מהשיטה התחתונה אם יצא במבוקש בלי חסרון ויתרון אז חשבונך מכוון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Examine and you will find [that it is true]. | ודוק ותמצא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Chapter Three: Subtraction |
שער החיסור | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If you want to subtract and deduct a number from a number, here is how you do that: | אם באת לחסיר ולנכות חשבון מתוך חשבון הילך איך לעשות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Write the number from which you wish to subtract and deduct above, according to its ranks, and write the number you subtract from it beneath, according to its ranks. | תכתוב החשבון שאתה רוצה לחסיר ולנכות ממנו אותו חשבון תכתוב למעלה והחשבון שאתה מנכה תכתוב למטה כפי מעלותיו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Then subtract it from the number above. | ואח"כ תנכה מן החשבון שלמעלה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Now, I shall write you an example to teach you: | ועתה אכתוב לך הדמיון להשכילך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה בקשנו לחסיר ט' מאות ותשעים ותשע מאלף | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הילך היאך תכתוב אותו כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נמצא הא' שבשיטה העליונה במעלה רביעי' עולה עשרה לגבי [ט'][30] שלישי' שבתחתונה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עתה נחסיר מאותו עשרה ט' וישאר [לך][31] א' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אותה א' תכתוב במקום גלגל השלישי נגד הט' שחסרת ואותו [ט'] [32] תמחוק כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עכשיו כמו כן הא' שבמעלה שלישי' שבשיטה העליונה עולה י' לגבי ט' שבשיט' תחתונה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
מאותו נחסיר ט' שנייה ונשאר א' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אות' א' תכתוב במקום הגלגל שני ותמחק ט' שנייה שחסרנו וגם תמחוק הא' העליונ' שבמעלה שלישי' שחסרנו ממנו ויהיה כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שוב נחסיר ט' תחת הגלגל אמ' שלמעלה במעלה שנייה כי גם היא עולה י' לגבי ט' כדלעיל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה כשנחסיר ט' מי' וישאר א' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אות' א' תכתוב במקום הגלגל ונמחוק הא' העליונה אשר במעלה שנייה וגם הט' נמחק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואז נשאר רק א' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נמצא כשחסירנו ט' מאות וצ"ט מאלף נשאר א' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דומיון [אחר אם][33] בקשנו לחסיר אב"ג מדה"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כתוב כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נחסיר ג' התחתונה מו' העליונה שכנגדה וישאר ג' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נמחוק הו' ונכתוב במקומה הג' הנשארת ונמחק כמו כן הג' [שנשאר][34] שלמטה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ויהיה כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שוב נחסיר ב' תחתונה [35]מה' עליונה שכנגדה וישאר ג' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אות' ג' נכתוב במקום הה' ונמחק הב' שלמט' [וגם ה' שלמעלה][36] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ויהיה כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שוב נחסיר א' תחתונה מד' עליונה [שכנגדה][37] וישאר ג'ג'ג' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
[ומחוק ד' שלמעלה וגם א' שלמטה וכתוב ג' במקום ד' שלמעלה][38] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
[ויהיה כזה][39] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נמצא כשחסרנו אב"ג מדה"ו לא נשאר כ"א ג'ג'ג' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
[וכאשר תמצא שהאות העליון אשר בקשנו לחסר ממנו פחות מן האות התחתון שתרצ' לחסר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כגון אם תרצ' לחסר זוח מן והט כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
היאך תחסור מרובה ממועט כזה ז' מן ו' לא תוכל וכן ו' מן ה' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
בדרך זה תעשה טול א' מן ה' אשר אצל ו' ומחוק הה' וכתוב ד' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אותו א' כמה עולה לחבירו עם הו' י"ו כי היא במעלה שנייה מן הו' הרי י"ו חסר ז' אשר בטור השפל מן י"ו וישאר ט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אחר כך צריך לחסר ו' מן ד' עשה ג"כ כמו שצויתיך מחוק א' מן ט' ויהיה במעלה שלפניו י"ד וחסר ממנו ו' וישאר ח' ול"ד זה שייך לשער הכפל קטון][40] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
[41]אשכילך היאך תעשה כי לא תוכל לחסר ז' מן ו' וכן ו' מה' בדרך זה תעשה בתחילה חסר ח' תחתונה מט' עליונ' וישאר א' מחוק הט' וכתוב א' במקומ' גם ח' תחתונ' מחוק ויהיה כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עתה צריכין אנו לחסר ו' תחתונ' מה' עליונ' ולא יכולנ' לכן לך לך אל הא' אשר לשמאל ה' עליונ' וצרפ' יחד א' וה' ויהיה ט"ו ונחסור הו' ממנו וישאר ט' מחוק הא' העליונ' גם ה' העליונ' וכתוב במקומם ט' גם ו' תחתונ' תמחוק וישאר כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שוב צריכין אנו לחסר ז' תחתונ' מו' ולא יכולנ' עתה לך לך וקח אחד מן הט' שבצד הו' בשיט' עליונ' וצרפ' יחד הו' גם הא' והיא עשרה אצל הו' ויהיה י"ו ועתה חסר ז' מן י"ו וישאר ט' מחק ו' ט' עליונ' וכתוב ט' ח' במקומם כאשר ציויתיך ומחוק ג"כ הז' התחתונ' ויהיה כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The procedure is complete, look carefully. | ותשלם המלאכה עיין היטב[42] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Checking Methods |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תרצה לידע אם אמת חשבת אם לאו אז תחבר שער החיבור א'ב'ג' עם ג'ג'ג' ואם תמצא ד'ה'ו' אז החיסור מכוון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
[ואם תרצ' לידע אם אמת חשבת אז חבר בשער החיבור ז'ו'ח' עם ט'ח' הנשארי' ואם תמצא ו'ה'ט' אז כיוונת][43] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| This is the rule: the subtraction chapter is the scales for the addition chapter and the addition chapter is the scales for the subtraction chapter. This is enough for the one who understands. | זה הכלל שער החיסור מאזני צדק לשער החיבור ושער החיבור מאזני צדק לשער החיסור ולמבין די | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Chapter Four: Multiplication |
שער הכפל גדול | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם בקשנו לכפול רל"ד ואלף על ד' אלפי' ושכ"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הילך צורתו כאשר תכתבנו חשבון האחד בצד השני ומכוון אות ראשון בחשבון תחתון תחת אות אחרון שבחשבון העליון כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נכפול א' תחתונה על ד' עליונה פי' נחשוב א' פעמי' ד' זה רק ד' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אות' ד' נכתוב נגד א' תחתונה ונמחוק א' תחתונה כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד נכפול ב' תחתונה על ד' עליונה ויעלה ח' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אות' ח' נכתוב נגד ב' התחתונה ונמחק ב' התחתונה כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד נכפול ג' תחתונה (על ד') על ד' עליונה ויעלה ב"א פי' י"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עתה נכתוב הב' נגד ג' תחתונה והא' על הח' אחריה ויעלה ט' על כי הוא מעלה אחת שהיא עשרה אחר הב' שלפניה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומחוק ג' התחתונה כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד נכפול ד' תחתונה על ד' עליונה ויעלה ו"א [פי' י"ו][44] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עתה נכתוב הו' נגד ד' התחתונה ובמקום ד' עליונה כי עתה נחשבנו הד' העליונ' בכל חלקיה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונכתוב הא' על הב' שאחריה ויהיה ג' כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומעתה נמשך ד"גב"א מעלה לאחורית תחת ג' העליונה כדי לחשוב הג' [עליונה][45] גם בכל תוצאותיה דגב"א כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נכפול א' תחתונה על ג' עליונה פי' ג' פעמי' א' זהו רק ג' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ושים אות' ג' על ט' שעל הא' התחתונה ויעלה ב"א פי' י"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עת' נכתוב ב' במקום ט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וא' שהיא עשרה שים אותה במעלה שנייה דהיינו בתוך הד' ונעשה הד' האחרונה ה' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונמחק הא' התחתונה כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד נכפול ב' תחתונה על ג' עליונה ב"פ"ג היינו ו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ושים אות' ו' על ג' שעליה ויהיה ט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונמחוק ב' תחתונה כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד נכפול ג' תחתונה על ג' עליונה ג'פ"ג ט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ושים אות' ט' על הו' שעליה ויעלה ה'א' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הה' תכתוב במקום הו' והא' במעלה שנייה דהיינו אל הט' שאחריה ויהיה י' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אך אין ראוי לכתוב י' כי אין [46]כותבין בחשבונות הציפרא י' רק עד ט' לכן נכתוב גלגל כזה 0 במקצוה ונכתוב א' במעלה של אחריה דהיינו אל הב' ועתה יהיה ג' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונמחוק ג' תחתונה כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד נכפול | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עתה נכתוב הב' במקום הג' העליונה כי נחשבנו ג"כ הג' בכל חלקיה והא' נכתוב במעלה שנייה אל הה' ויהיה ו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונמחק ד' תחתונה כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועתה נמשיך דגב"א מעלה אחת לאחוריו תחת הב' העליונה כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נכפול א' תחתונה על ב' עליונה א"פ"ב היינו ב' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אותה ב' נכתוב נגד א' תחתונה במקום הגלגל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונמחק א' תחתונה כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שוב נכפול ב' תחתונה על ב' עליונה ב"פ"ב' היינו ד' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אות' ד' נכתוב למעלה נגד ב' תחתונה דהיינו אל הו' הרי י' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כתוב במקום הו' גלגל ואחר הגלגל [תוסי]ף א' דהיינו אל הב' ויהיה כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שוב נכפול ג' תחתונה על ב' עליונה ב"פ"ג היינו ו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אות' ו' נכתוב נגד ג' תחתונה דהיינו אל הב' [עליונה][47] ויהיה ח' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונמחק הג' התחתונה ויהיה כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שוב נכפול ד' תחתונה על הב' העליונה ב"פ"ד ח' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נכתוב ח' במקום ב' עליונה כי גם [חשבנו][48] הב' בכל חלקיה ונמחק ד' תחתונה כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נמשך דגב"א עוד מעלה אחת לאחוריו כדי לכפול אות' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נכפול א' תחתונה על א' עליונה[49] א"פ"א היינו א' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אותה א' נכתוב נגד א' התחתונה במקום הגלגל ונמחק א' תחתונה כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שוב נכפול ב' תחתונה על א' עליונה ב"פ"א היינו ב' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אות' ב' נכתו' על הב' התחתונה דהיינו אל הח' נוסיף ב' על הח' ויהיה י' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כתוב במקום הח' גלגל ואחר הגלגל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נוסיף א' על הא' ויהיה ב' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונמחק ב' התחתונה כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שוב נכפול ג' תחתונה על א' עליונה ג"פ"א היינו ג' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אות' ג' נכתוב נגד ג' תחתונה דהיינו ח' הרי י"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נמחוק הח' ונכתוב במקומה א' ואחריה במקום הגלגל א' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונמחק ג' תחתונה כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שוב נכפול ד' תחתונה אל א' עליונה ד"פ"א היינו ד' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אות' ד' נכתוב במקום א' שכנגדה אבל לא נצטרף אות' א' עם הד' כמו שצויתיך כבר כי על אות' א' כפלנו עכשיו [דגב"א ול]א היתה הא' רק לסימן כמה פעמי' לכן לא חדייה לצירוף | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונמחק ד' תחתונה כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נמצא כי אבג"ד פעמי' דגב"א עולה בחשבון ד'א'א'ב'ג'ג'ה' דהיינו י"ד ומאה אחת וב' אלפים ול"ג ריבואות וה' מאות ריבואות וד"ל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
[50]דומיון אחר הנה בקשנו לכפול ט' ומאתים על ג' אלפי' ול' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הילך היאך תכתוב צורתו כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נכפול ב' התחתונה על ג' עליונה ב"פ"ג היינו ו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אות' ו' נכתוב ב' נגד ב' תחתונה ונמחק ב' תחתונה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וגם הגלגל שלפני הב' התחתונ' מחוק וכתוב גלגל לפני הו' כזה כי נכפול גלגל פעמי' ויהיה גלגל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שוב נכפול ט' על ג' שעליה ט"פ"ג היינו כ"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואות' כ"ז נכתוב למעלה במקום הג' האחרונה ויהיה ז' במקום הג' וב' במקום הגלגל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונמחק ט' התחתונה כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נחזור ט0"ב לאחוריו ב' מעלות כדי שתהא ט' התחתונה שהוא אות ראשונה שבשיטה תחתונה נגד ג' עליונה כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וא"ת למה לא כתבם ט'0'ב' תחת הגלגל העליון ודאי עשינו כך אבל מ"מ לא עולה יותר מגלגל כי אם תמצא אותיות כתובות ואתה צריך לצרף עמהם גלגל אין צורפי' עמהם ול"ד כמו שפי' לקמן | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נכפול ב' התחתונה על ג' עליונה ב"פ"ג היינו ו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אות' ו' נכתוב נגד ב' תחתונה דהיינו אל הז' ויהיה י"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נמחק הז' ונכתוב במקומה ג' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואחריה נוסיף א' על ב' כי היא עשרה כמו שכתבתי לעיל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונמחק ב' וגם הגלגל שלפני הב' נמחק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואין אנו צריכי' לצייר אותו גלגל למעלה כמו שעשית כבר כי בלאו הכי יש גלגל כנגדו וכ"ש אם היה אות נגד הגלגל שלא היינו מוחק' האות לצייר שום גלגל שאם היינו מוחק' האות כדי לצייר שם גלגל אז היינו ממעטין החשבון ואם לעשות כדי להרחיק המעלה זה אינו כי בלאו הכי האות מרחיק המעלה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כללא דמילתא לעולם אין אנו מציירין גלגל התחתון למעלה אלא אם אין כנגדו שום דבר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אבל אם יש שום גלגל [או אות][51] נגד אותו גלגל התחתון אז אין אנו צריכין לצייר שום גלגל כי ציורו אינו אלא [שומר המעלה][52] כמו שכתבתי לעיל [כי הגלגל מודיע שאות אחריו עולה מעלה אחת יותר ממה שהיית עושה אם לא היית שם שום ציור גלגל][53] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועתה נחזור לחשבון דלעיל כשתעשה כמו שפירשתי אז יהיה כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שוב נכפול ט' תחתונה על ג' עליונה ט"פ"ג היינו כ"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נכתוב במקום ג' עליונה ז' ואח"כ במקום גלגל ב' ונמחק ט' תחתון וגם הג' העליונה [מח]ק כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נמצא שבמאתים וט' פעמי' ג' אלפי' ול' יש ס"ג רבבות וג' אלפי' ומאתים ושבעי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| This belongs to the chapter on multiplication. | [54]וזהו שייך לשער הכפל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| I will show you another way to multiply: | הנה אראך בדרך אחר לכפול | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם תרצה [לידע][55] כמה ט"ו פעמי' תתר"ף | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עשה טור ראשון מתתר"ף ושים תחתיו ט"ו ה' תחת הא' וא' לשמאלו כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אז תכפול הא' על כל הטור העליון ואח"כ ה' על כל הטור העליון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכה תאמר אפ"א הרי א' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שים א' כנגד א' השפל מן הכפלות כל כך גבוה שיהא הוא למעלה מן התתר"ף [מכוון כנגד הא' התחתונ' ויהיה צורת' כזה][56] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואח"כ כפול א' פעמי' גלגל הרי גלגל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שים גלגל לפני הא' אשר כתבתי למעלה מן התתר"ף [מכוונ' נגד הא' בשיט' ראשונ' ויהיה כזה][57] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואח"כ כפול א' פעמי' ח' יעלה ח' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כתוב ח' לפני ה0' שכתבת [בשיט' עליונ' מכוונת נגד הציפר' שבשיט' אמצעי' ויהיה כזה][58] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואח"כ כפול אפ"0 ויעלה 0' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שימהו לפני הח' אשר כתבת [בשיט' עליונ' מכוון נגד 0' שבשיט' אמצעי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
גם תמחוק הא' כי נכפל על כל התתר"ף][59] ויהיה כזה דמיונו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה כפלת הא' על כל התתר"ף | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואח"כ כפול הה' על כל התתרף | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותתחיל לכופלו כנגדו ממש למעלה כאשר התחלת לכפול הא' כנגד [א'][60] כן תכפול הה' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ה' פ"א הוי ה' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שים ה' נגד הא' למעלה הימנו דהיינו במקום גלגל [כזה][61] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואח"כ אמור הפ"0 | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם לא היה נכתב מאומה על הגלגל היית נותן שם גלגל עכשיו שרשום עליו ח' לא תעשה מהגלגל כלום כי אוחז מקומו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואח"כ אמור הפ"ח יעלה ארבעים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולא תשימהו במקום הגלגל כי תצטרך לגלגל אלא כתוב ד' על הח' לשמאל אז תמצא ד"ח זה על זה תמחוק שניהם ותכתוב במקומו ב' ועל הה' אשר כתבת לשמאל כתוב א' הרי ו' [כזה][62] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואח"כ אמור ה"פ גלגל היינו גלגל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שים גלגל על גלגל ויהא דמיונו כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תבין שלא נשלם הטור העליון נגד הטור השפל עד אשר תכפול כל האותיות מן הכפולות על כל החשבון ול"י סליק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Checking Methods |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If you are in doubt saying who said this is so, here are scales to check whether you were wrong or not: | [63]ואם לבך מפקפק לומר מאן יימר שכך [הוא][64] הילך מאזנים היאך תשקול ותבחין אם טעית אם לאו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
בראשונה תקח חשבון שבשיטה העליונה אשר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם לא תמצא אפילו פעם אחת [ט'][66] אז תקח הכל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואח"כ תיקח החשבון התחתונה והשלך ג"כ אז בט"ט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומה שישאר בידך קח נא והשלך אותו בשער הכפל על מה שנשאר לך בשיטה עליונה אחר שהשלכת ממנו ט'ט' ומה שיעלה בידך השלך אותו ג"כ בט"ט ומה שנשאר שלא הגיע לכלל ט' קח נא בידך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואח"כ קח החשבון היוצא לך כבר כשכפלת הב' חשבונות יחד והשלך אותו ג"כ בט"ט ט"ט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומה שנשאר בידך אם הוא מכוון כנגד מה שנשאר לך כבר ידוע תדע שיפה חשבנת ואם לאו בודאי טעית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועתה נשקול חשבון דלעיל למען יבין המבין ויוסיף לקח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הדמיון היה 0"ג0"ג פעמים ט0"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
בתחילה נחבר ב' פעמי' ג' היינו ו' ואין כאן אפילו פעם אחת ט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואח"כ נחבר חשבון התחתון דהיינו ט0"ב נחבר ט'ב' היינו י"א השלך ט' וישאר ב' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נכפול ב' על ו' השלך ט' וישאר ג' וזה יהיה לך לזכרון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
זה הכלל ראה בכפל מה נשאר לך כמו עתה הוצרכנו לכפול בפ"ו והיה י"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואח"כ ראה מה שיעלה בידך כשכפלת 0'ג'0'ג' על ט'0'ב' דהיינו 0'ז'ב'ג'ג'ו' נחבר אותם יחד ויהיה כ"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Chapter [Five]: Multiplication in a Table [Gelosia] |
שער הכפל בסולם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| This diagram is called a table [lit. ladder] and it is appropriate for the multiplication chapter. | ציור זה נקרא סולם ויפה לשער הכפל [גדול][67] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| As the size of the numbers you want to multiply by each other, draw the number of cells. | וכמה שתרצה לכפול חשבון על חשבון כן עשה מניין הבתים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם תכפול חשבון בג' מעלות על ג' מעלות כמו כן תצייר שליבות הסולם ג' בתים לארכה ולרחבה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תכפול סך בג' מעלות על ב' מעלות עשה ג' בתים לרחבה וב' בתים לארכה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן להפך מלמעלה למטה אחד המרבה ואחד הממעיט ובלבד שיכוון לבו כל אות' [במקומו][68] לפי הציור | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון אם תרצה לידע כמה כ"א וג' מאות פעמי' נ"ד ות"ר שרשימתו אב"ג דה"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
רשום למעלה על הבתים כאשר תראה בסולם שרשמתי והנכפול כתוב בצדו זה תחת זה כמצוייר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כי כל בית נחלק מחציתו העליון אחדים והתחתוני' עשירים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואח"כ חשוב ד' פעמי' ב' ויעלה ח' רשמיהו בחצי בית העליון שתחת ב' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואח"כ חשוב כמה ד'פ'ג' ויעלה ב'א' רשמיהו באותו בית שתחת הג' וכתוב ב' בחצי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומעתה תמחוק הד' כי היא נחשבת בכל חלוקיה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
מעתה תצטרך לחשוב הפ"א ויעל' ה' ורושומיה בבית שבצד הה' מכוון תח' הא' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואח"כ חשוב הפ"ב ויעלה 0"א רשומיהו בחציי התחתון בבית חמישין | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואח"כ חשוב הפ"ג ויעלה ה"א רשומיה בבית שישי [ה' בחצי העליון א' בחצי תחתון][70] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומעתה תמחוק הה' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה חשוב ופ"א יעלה ו' רשימהו בצידו מכוון בטור שתחת הא' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ו' פעמים ב' ב"א רשימיהו בבית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
[ו' פ"ג היינו ח"א רשימיהו בבית האחרון][71] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה כפלנו כולו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואשכילך כמה יעלה סכום שלה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
קח אות ראשון שבבית ראשון שהיא ד' ותכתביהו במקום מיוחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואח"כ חשוב בשליבה שנייה בכל השיפוע מאות ח' עד ה' וצרוף הה' אל הח' ויעלה ג"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הג' כתוב בצד הד' הנרשמת כבר כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והא' שהיא עשרה צרוף לשליבה שתחתיו וחבריהו אל הב' בתחיל' שליבה שלישית ויעלה ג' וחבר אח"כ גם הו' שבסוף השיפוע ויהיה ט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ורשמיהו אצל ד"ג כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואח"כ חשוב וצרוף אותיות שבשליבה רביעי' מראש' ועד סופ' ויעלה ט' וכתביהו בצד דג"ט כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה חשוב אותיות שבשליבה חמישית בשיפועו ויעלה צירופו עשרה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכבר אמרתי שלעולם העשירי' מצטרפ' לשליבה שתחתיו ואין רושמי' אותה בצד המיוחדי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אבל תצטרך להשי' גלגל במקום העשרה לשמור המעלו' הואיל ואין נשאר אפילו אחד [לשמור][72] המורה המעלה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה כשתצטרף העשרה לא' שבשליב' אחרונה יעלה ב' וכתביהו בצד דגט"ט המיוחדי' כזה ד ג ט ט 0 ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
זה מכוון חשבון של כפולתם אב"ג על דה"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומאזנים שלו כמו שכתבתי לעיל בשער הכפל [הגדול][73] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דו"ד פעמי' דו"ד יעלה חשבונו וט"בהא"ב ודו"ק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The multiplication chapter is complete. | סליק שער הכפל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Multiplication - Check |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| When you want to know the scales, if the result is correct, when you have the product: | [74]כשתרצה לידע משקל אם אתא שפיר כשיש לפניך הכפל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כגו' א דוד פעם דוד כגו' ו'ט'ב'ה'א'ב' או כל כפלים אם הן שפיר או לאו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אז קח לפניך דוד במניין נמצא י"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואמ' כמה הוא יותר מט' נמצא ה' ותשליך הט' ממך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואמ' כמה ה' פעמי' ה' נמצא כ"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותשליך ממך כל הט' שבתוך כ"ה ויתר לך ז' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אז תשליך נמי ו'ט'ב'ה'א'ב' בט' אז ישאר לך ז' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Do the same with all the products in the world. | וכן תעשה לכל הכפולים שבעולם ודו"ק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Chapter Six: Division |
[75]שער החילוק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The chapter on division is appropriate for dividing a large number into a few smaller numbers | שער החילוק והוא טוב לחלק חשבון גדול לכמה חשבונות קטנים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| You should know that in this chapter the first digit of the bottom row is not written corresponding to the last digit of the top row, as in the multiplication chapter, but the top row is written according to its ranks and the bottom row [is also written] according to its ranks, so that the last digit of the bottom row corresponds to the last digit of the top row. | ועתה ידוע תדע שאין כותבין בשער זה כמו בשער כפל אות ראשונה משורה תחתונה נגד אות אחרונה משורה עליונה אלא כותבין שורה עליונה כפי מעלותיה ושורה תחתונה כמו כן כפי מעלותיה ויכוין אות אחרונה שבשורה תחתונה נגד אות אחרונה שבשורה עליונה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If the last digit of the bottom row [= divisor] is greater than the last digit of the upper row [= dividend], the bottom row is shifted one rank backwards, unless the bottom row has more than one digit. | [ואם אות אחרונה שבשורה תחתונה יותר מאות אחרונה שבשורה עליונה][76] אז יסוג שורה תחתונה מעלה אחת לאחוריה [אלא א"כ דאותו שורה תחתונה יש בה יותר מאות אחת][77] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If they [= the last digits of the two rows] are the same [the bottom row] should not be shifted, unless it has more than one digit. | אבל אם עלו שוות לא יסוג לאחריה אלא [78]א"כ דאותו שורה יש בה יותר מאות אחת[79] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| When it has more that one digit, the whole row should be shifted one rank backwards, so that the upper digits can be divided by the lower digits. | וכשיש בה יותר מאות אחת יסוג כל השורה מעלה אחת לאחוריה ואז יכול לחלק אותיות עליונות על אותיות תחתונות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The quotient is written corresponding to the first rank of the lower row, whether this rank is a non-zero digit, or a zero. | והחילוק שחילקו יכתוב נגד מעלה ראשונה שבשורה תחתונה הן אם אות' מעלה אות או גלגל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| When you divide by 2 digit, meaning that there are 2 digits in the bottom row, both are [subtracted] the same [number of times], whether [this number consists of] 3 digits, or 4 and so on endlessly, as the number of times you take the last digit of the number, so is the number of times you take the rest of the digits. | וכשתחלוק ב' אותיו' פי' שהיו בשור' תחתונה ב' אותיות אז אין חילוק של שתיהן רק מניין אחד [יהיה][80] ג' אותיו' או ד' וכן עד סוף כל הדורות כל כמה פעמים שלקחת האות האחרונה מן החשבון כך תקח כל שאר האותיות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| This is enough to understand; examine and you will find [that it is true]. | ול"ד ודו"ק ודו"ק ול"ד וד"ל ול"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Here is the chapter of division, suitable for everything and also for dividing a large number into several small numbers. | והילך שער החילוק וטוב לכל דבר וכן עמ' דבר לחלק חשבון גדול לכמה חשבונות קטנים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Examples | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| I shall write examples so that one will understand. | ועתה אכתוב דמיונים למען יבין המבין | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Instruct a wise man, and he will become wiser... and increase in learning [Proverbs 9, 9]. | ותן לחכם ויחכם עוד ויוסיף לקח[note 5] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Now I start dividing by a small number: | ועתה אתחיל בחילוק קטן | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם ישאל השואל כמה פעמי' ז' יש במאתים וי"ח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הילך היאך תעשה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תכתוב המאתים וי"ח ויהיה כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והז' היה מן הדין לכתוב תחת הב' כדי לחלק הב' לז' ז' פי' למנה כמה פעמים ז' יש בב' אלא שאין הב' מגיע לחילוק של ז' לכן נסוג הז' לאחור כדי שתהא הב' במעלה שנייה [לה][81] ותעלה לנגדה עשרים ואחד כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואותן עשרים ואחד תחלוק לז' ז' פי' מנה כמה פעמי' ז' יש בעשרי' ואחד ונמצא שיש בה ג' פעמי' ז' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואותה ג' כתוב למעלה על הא' שבשורה עליונה נגד ז' שבשורה התחתונה כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואח"כ תמחוק הא"ב שמצאת בהם ג' פעמי' ז' ואח"כ יסוג הז' מעלה אחת לאחוריה ויהיה תחת הח' כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומנה כמה פעמי' ז' יש בח' ולא נמצא בה רק פעם אחת ז' ואחד עודף | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אז תכתוב א' נגד הז' על הח' כזה והאחד העודפת כתוב במקום הח' כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נמצא ברי"ח יש ל"א פעמי' ז' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן לעולם ועיין[82] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דומיון [אחר][83] יש לי עשרת אלפים בצן ומאה ועשרים ושבע בצן ואבקש לידע כמה זהובי' בתוכו שכל אחד ט"ו פצן | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה רשמהו כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה תרשום תחת זה ה"א ואם תכתבנו תחת הסופיים כמחוייב נמצא א' תחת א' ולא ישאר לך דבר וא"כ מאין תקח אפ"ה כי כמה [84]פעמים שתקח אות אחת כמו כן תקח חבירתה כמו שכתבתי לעיל וא"כ נסוג [ה'א'][85] אחור ונכתוב הא' תחת [גלגל וכתו' ה' תחת א' שלפני גלגל ויהיה][86] כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה צריכין אנו לשער באם נקח הא' מהאותיות שעליו שגם יעדיף למצא הה' בהעודף בחשבון הזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תרצה לומר ליקח ט' פעמי' מעשרה א"כ לא ישאר רק א' וחברו במקום הגלגל [ויהיה א"א][87] ומעתה לא תוכל ליקח טפ"ה מא"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן אם תקח ח' פעמי' ב' מן א"ב [וכן אם תקח ח' פעמים א' לא ישאר רק ב' ולא תוכל ליקח ח' פעמים ה' מן ב"א][88] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן אם תקח ז"פ [מעשרה לא תוכל ליקח חפ"ה][89] לא ישאר לך אלא א'ג' ולא תוכל ליקח ז' פעמים ה' מן א"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אלא תקח ו' וישאר ד' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואז כתוב [ו'][90] על הא' [ויהיה][91] כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואז תוכל ליקח ו' פעמי' ה' מן א'ד' וישאר לך מן הד' שהיא א' עשרה ואח"כ כתוב א' במקום הד' [ומחק ה' ויהיה][92] כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואח"כ בקשנו ליקח הא' מן החשבון כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
י' פעמי' לא תוכל ליקח כמו שפירשתי וגם זה הכלל נקוט בידך לעולם לא תוכל ליקח י' רק ט' או ח' או ז' [ולמטה][93] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
לכן עשה כך כתוב הא' תחת ב"א עליונה כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונקח ז' פעמי' נשאר ד' שעודף י"א על ז' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונכתוב ז' למעלה כנגד הה' תחתונה ונמחק הא' תחתונה וכנגד ב' עליונה ונמחק ג"כ הא"א עליונה ונכתב תחתיהן ד' כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה נקח ז' פעמי' ה' מן [ד'][94] שבצידה דהיינו ל"ה ויעדיף חמשה כי הד' היא במעלה שנייה מן הה' [עולה][95] ארבעי' ועתה לקחנו ממנו ל"ה נשאר ה' צרוף הה' אל הב' שלפניה ויהיה ז' כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה נכתב ה'א' תחת ז'ז' העודף כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונקח ה' פעמי' א' מז' [כי ז' פעמים לא נוכל ליקח כמו שפי' לעיל שאז לא נוכל ליקח][96] ז' פעמי' או ו' פעמי' ה' ודו"ק לכן ניקח ה' פעמי' ונכתב ה' למעל' בצד הז' [נגד ה' תחתונה][97] ויעדיף ב' [אותו ב' כתוב במקום ז'][98] כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה ניקח ה' פעמי' ה' מז"ב ויעדיף ב' שאינו מגיע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נמצא מכוון שעשרת אלפי' ומאה וכ"ז [פצין עולה ה' זהו' וגם ת"ר זהו' ושני פצין][99] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Checking Methods |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If you have doubts multiply the last [result] by 15 and you will receive the original number you asked about. | ואם לבך מגמגם כפול העודף האחרון על ה'א' ויצא לך חשבון הראשון ששאלת | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| This is the scales of the division chapter. | וזהו מאזני צדק על שער החילוק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| We find that the multiplication is verified by division and the division is verified by multiplication. | נמצא כפל מבורר החילוק והחילוק מברר הכפל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון למאזנים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כתוב ה'א' וכתוב תחתיו הו"ז הנשאר באחרונה חוץ מהב' שלא הגיע לחשבון ט"ו אל תצטרף עמהם עד סופו חברה לאות ראשונה כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפול ו' על א' יעלה ו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפול ז' על א' יעלה ז' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפול [100]ה' על א' יעלה ה' כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה כתוב הז"ו תחת ה' של ה"א כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפול ו' על ה' יעלה ל' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חבריהו לז' שעליו כמו שציויתיך בשער הכפל א"כ צריך אתה ליתן הג' אל הו' ויהיה ט' כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואח"כ כפול ז' על ה' ויעלה ה"ג וה' דהיינו 0"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עשה גלגל במקום ה' ותן ד' לז' ויהיה א'א' כתוב א' במקום ז' ותן א' אל הט' ויהיה י' אז צריך אתה לעשות במקום הט' גלגל ואחריו א' כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואח"כ כפול ה' על ה' ויעלה ה"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כתוב ה' במקום ה' העליונה ואל תצרף ה' ראשונ' עליה כמו שציויתיך בשער הכפל ודו"ק ואח"כ כתוב ב' במקום גלגל כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה חבר ב' שלא הגיע לכלל ט"ו אל הה' הראשונה ויהיה ז' ואז יהיה החשבון כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והיינו כיון השאלה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| With God's help, the chapter of division is complete. | בע"ה סליק שער החילוק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
[101]ועוד אראה לך דומיון חושבתי מן חו"ה פעמי' חו"ה הכי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ורציתי לחלק אם חושבתי שפיר וכה תעשה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תכתוב לפניך החשבון שעולה ממנה ותכתוב תחתיו חו"ה וצורתו כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כי לא אתה יכול לחלק ה' מן ג' כי ג' לא מגיע אפי' פעם אחת לה' לפיכך סוג הה' לאחרי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואז תאמר כמה פעמים ה' בב"ג זהו ה' פעמים אע"ג שיש לי לקח עוד פעם אחת ה' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אלא מטעם זה אני לקח נוטל אות' ה' פעמים ולא יותר כי אם אני נוטל ו' פעמי' אז לא היה נשאר מן הב"ג אלא ב' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואז לא היה יכול לקח הו' מן חו"ה נמי ו' פעמי' מן ב"ב פי' מן כ"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והדין בזה השער כי כמה פעמי' שתחלק האות ראשו' אז אתה עושה לכל האותיות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
לפי זה אני נוטל ה' פעמי' ואז נשאר ז' ואז אני יכול לנטול גם הו' ה' פעמים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואות' ה' תכתוב למעלה על הו' נגד הח' התחתונ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונמחוק הב"ג ותכתוב במקומ' הז' וצורתו כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ושוב תחלוק הו' מן חו"ה נמי ה' פעמים מן ב"ז ואז נשאר לך ב"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותמחוק הז' ותכתוב במקו' ד' וצורתו כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואז תחלוק הח' נמי ה' פעמי' מן וב"ד פי' כ"ו ד' מאות אז תקח מאה מן הד' מאו' ועוד נשאר ג' מאות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואז קח מן המאה הח' פעם ה' וזהו 0"ד פי' מ' ועוד נשאר 0"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואותו ו' תשים על הב' ויהיה ח' וצורתו כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נמשוך חו"ה לאחריה תחת הב' וצורתו כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואז תאמר כמה פעמי' ה' בח"ג ויעלה ו' ועוד נשאר ח' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותמחוק הג' שאחר הח' והו' העולה מן הח"ג תכתו' על הב' בשור' עליונ' נגד הח' שלמטה וצורתו כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואז תקח הו' נמי ו' פעמי' מן ו"ח פי' מן פ"ו ועשה הכי תקח ה"ג פי' ה"ל מן ה"ח ואז נשאר נ' וקח הו' שלפני הח' ושים במקומ' גלגל כי ו' פעמי' ו' זהו ו"ג וצורתו כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותמחוק הו' של חו"ה כמו שציירתי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ושוב קח הח' נמי ו' פעמים מן ה' מאות ואז שוב עשה הכי קח מאה מן הה' מאות ועוד נשאר ד' מאות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וקח מן המאה ח' פעמי' ו' וזהו ח"ד פי' מ"ח ואז קח מן המאה נ' ואז נשאר נ' ואות' נ' תכתו' במקו' הגלגל שלפני הד' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועוד יש ב' יותר שאני צריך אלא מ"ח ואני לקחתי נ' ואות' ב' תשים על הב' של אחר הד' הראשונ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונמחוק הח' מן חו"ה וצורתו כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נמשוך חו"ה לאחריה תח הח' תחת הד' והו' תחת הד' השניי' והה' תחת הה' וצורתו כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואז תאמר כמה פעמי' ה' בה"ד פי' מ"ה ויעלה ח' פעמי' ואות' ח' תכתו' למעלה על הד' נגד ח' התחתונ' ועוד נשאר ה' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותמחוק הד' שאחר הה' ותמחוק הה' של חו"ה וצורתו כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ושוב נחלק הו' נמי ח' פעמים מן ד"ה פי' מן נ"ה וע ועשה הכי קח מן הנ' ח' פעמי' ו' ועוד נשאר ב' כי ח' פעמי' ו' זהו ח"ד פי' מ"ח ועוד נשאר ב' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואות' ב' תשים על הד' שלפני הה' והוה ו' וצור | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונמחק ו' של חו"ה והה' אחר הד' וצורת' כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואז תאמר ח' פעמי' ח' וזהו ד"ו פי' ס"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
סליק דומיון של שער החילוק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נמצא כשחלקתי חו"ה מן ד'ב'ו'ב'ב'ג' אז לא נשאר מאומה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Likewise, whenever you multiply by the same thing, as 568 times 568, or 464 times 464, then you divide by what you multiplied and you have nothing left, the calculation is correct. | וכן בכל מקום אשר אתה מחשב דבר השוה כגו' ח'ו'ה' פעמי' ח'ו'ה' או דו"ד פעמי' דו"ד וכשתחלק ממנ' אות' שחשבת ולא נשאר לך מאומה אז החשבון אמת ודו"ק ותמצא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועוד אראה לך דומיון שאינ' חשבון שוה כגו' א"ב פעמי' ה"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואז אתה לא עושה כדרך זה קח חד מן הפרטות הא"ב או הה"ד ותחלק מן החשבון ואז עולה הפרט השיני ודו"ק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
[102]דומיון חשבנו ה"ד פעמים א"ב ועולה ממנ' כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואז נחלק אם חשבנו אמת | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אז קח הכלל העולה ותכתוב תחתיו חד פרט ותאמר כמה פעם זה הפרט בזה הכלל אז עולה הפרט השני' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והילך צורתו אשר תכתביהו הכלל ותחתיו חד פרט איזה שתרצה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועת' נרא' קח הכלל ה'ד'ט' ותכתוב תחתיו הפרט ה"ד וצורתו כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותאמר כמה פעמים ד' בט' נמצא ב' פעמי' ולא יותר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אות' ב' תכתוב על הד' נגד הה' שלמט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותמחוק הט' ותכתוב במקומ' א' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואז קח הה' נמי ב' פעמי' מן ד"א ונשאר הד' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונמחוק הא' והה' שלמט' וצורתו כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואז תמשוך הה"ד מעלה אחת לאחריה הה' תחת הה' והד' תחת הד' וצורתו כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואז תאמר כמה פעמי' ד' בד' נמצא רק א' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואות' א' תכתו' על השני ה' קודם הב' וצורתו כזה ונמחק השני ד' כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נמצא כשחלקתי חד פרט אז עולה הפרט השיני | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן תמצא בכל פעם אשר אתה מחלק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
[103]והילך שער טוב לראות אם חלקתי אמת וטוב מאד מאד ואראה לך דומיון רציתי לחלק כמה פעם ז' בב' מאות וי"ח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
קח הז' שחלקתי ושים אותה תחת הג' [כזה] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותאמר כמה ז' פעמים ג' זהו כ"א א"ב פי' כ"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואז קח הז' ושים אות' תחת הא' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותאמ' ז' פעמי' א' זהו ז' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואות' ז' תכתו' קודם הא"ב שעולין מן ג' פעמי' ז' כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואז קח הא' שלא חלקת לז' ושים עתה על הז' שעולה השת' מן א"ג פעם ז' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נמצא כשלקחתי הח'א'ב' וחלקתי ממנ' כמה פעמי' ז' ועולה א"ג ונשאר א' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואז לקחתי הא"ג וזרקתי לתור הז' וחשבתי כמה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואז לקחתי הא' שנשאר כבר וזרקתי על זה הז' והוה ח' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נמצא ח'א'ב' כמו בראשון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Chapter Seven: Proportions |
[104]שער עירוכין | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| This respectable chapter is called the chapter on proportion, which according to the general opinion is needed in order to count, to calculate, to make wise, to educate, to know and understand a thing from a thing, to evaluate a thing after thing, and to comprehend its foundation and its nature. | שער הנכבד ונקרא שער הערך אשר לכולי עלמא צריך למנות ולחשוב ולחכם ולהשכיל לדעת ולהבין דבר מתוך דבר ולהעריך דבר אחר דבר ולעמוד על יסודו ועל בוריו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If you buy a large amount of goods for a high price and you need to estimate a small amount of goods according to this price, meaning how much should cost the small amount of goods you bought according to this price? | כיצד אם לקחת מקח גדול בדמים מרובים ואת צריך להעריך מקח קטן לפי אותה דמים פי' כמה מגיע לפי אותה הדמים למקח הקטן שקנית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Or vice versa, you buy a small amount of goods for a low price and you want to estimate a large amount of goods accordingly. | או להיפך שקנית מקח קטן בדמים מועטי' ואתה רוצה להעריך אחריו מקח גדול | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Here is how you calculate, count, and evaluate: | הילך היאך תחשוב ותמנה ותעריך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Write the large amount of goods and write the price of the large amount of goods beneath the large amount of goods. Then, write the small amount of goods beneath the price. | תכתו' המקח גדול וסכו דמים מן הגדול המקח תכתוב תחת המקח גדול ואח"כ תכתוב המקח הקטן תחת הסכום | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Multiply the price of the large amount of goods you wrote above, below the large amount of goods, by the small amount of goods. Take the product and divide it, according to the chapter of division, by the large amount of goods; what you receive is the price of the small amount of goods. | ותכפול דמי המקח הגדול אשר כתבת למעלה אחר המקח הגדול תכפול אותו על מניין מקח קטן והעולה קח בידך ותחלוק אותו בשער החילוק על המקח הגדול ומה שיעלה בידך כל כך ערך המקח הקטן | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If you are left with fractions that are less than the divisor, say: I have fractions left of which a certain amount is one unit of the required. | ואם יותר לך חלקים שלא יעלו לחלק המבוקש אז תאמר עוד נשאר לי חלקים כך וכך שסכום כך וכך עולה לחלק אחד של המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Or vice versa, you want to evaluate [the price of] a large amount of goods according to [the price of] a smaller amount of the goods: write the small amount of goods above, then write the known price of this amount of goods and write the large amount of goods below, beneath the price of the small amount of goods. | ואם להיפך שאתה רוצה להעריך חשבון מקח גדול אחר חשבון מקח קטן אז תכתוב חשבון הקטן למעלה ואח"כ תכתוב דמים הידועים לאותו מקח והחשבון הגדול תכתוב למטה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Multiply the large amount of goods by the known price of the small amount of goods, and divide the product, according to the chapter of division, by the small amount of goods above as above. | ותכפול חשבון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| This is rule [of the calculation procedure]: | וזה הכלל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| First, write the amount of goods you know. Then, write its price, and after it the amount of goods, [whose price] you wish to know and evaluate. | המקח אשר ידעת תכתוב תחילה ואח"כ תכתוב דמיו ואח"כ המקח אשר בקשת לידע להעריך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Multiply, then divide as I have instructed you. | ותכפול ואח"כ תעריך כמו שציויתיך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Word Problems |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Now I will write for you two or three examples, so you may understand and become wise. | ועתה אכתוב לך ב' או ג' דמיונות כדי שתוכל להבין ולהשכיל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Pricing Problems - Find the Price |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
איש אשר ישאלך הנה קניתי ע' אמות בגד עבור מ' דינרים כמה מגיע לנ"ה אמות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אז תכתוב תחילה הע' אמות ואח"כ דמי הע' אמות ואח"כ הנ"ה אמות אשר בקשת לידע ערכיהן כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותכפול נ"ה על מ' פי' תמנה ותחשוב כמה עולה נ"ה פעמי' מ' והעולה הוא ב' אלפי' וב' מאות כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואח"כ תחלק ב' אלפי' לע'ע' פי' חלוק בשער החילוק כמה פעמי' ע' יש בהן והחילוק הוא ל"א פעמי' ע' ול' חלקי' קטני' שע' הם חלק אחד דהיינו דינר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נמצא השאלה ששואל לך כשקוני' ע' אמו' עבור מ' דינרי' כמה מגיע לנ"ה ע' אמות השב לו מגיע לו ל"א דינרי' ועוד ל' חלקי' שע' מהם עושה חלק שלם דהוא דינר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תעלה על דעתך מאן מוכח ומאן לימא לן דכך הוא שמא הערך אינו כמו שחשבת | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אז צא וחשוב הט"ו אמות העודפי' על הנ"ה כי מנ"ה עד ע' יש ט"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותעריך כמה מגיע לט"ו אמות כשע' באות עבור מ' דינרי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והילך היאך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תכתוב הע' אמות למעלה ואח"כ למטה הימנו המ' דינרי' ואח"כ הט"ו אמו' כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותכפול הט"ו על המ' פי' חשוב כמה עולה ט"ו פעמי' מ' ועולה ו' מאות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואח"כ תחלוק ו' מאות לע' ע' פי' תמנה כמה פעמי' ע' ישנו בו' מאות ויעלה שמנה פעמי' ע' ועוד ישארו מ' חלקי' שע' מהן עושי' חלק שלם פי' דינר אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נמצא כשקנית ע' אמות עבור מ' דנרים מגיע לט"ו אמו' ח' א' דנרים ומ' חלקי' שע' מהם עושי' חלק שלם דינר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולמעלה חשבנו שמגיע לנ"ה אמות ל"א דינרי' ול' חלקי' שע' מהן עושי' חלק שלם פי' דינר אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עתה חבר הערך יחד ל"א דינרי' וח' דינרי' הרי ל"ט דינרים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומ' חלקי' הנותרי' בט"ו ול' חלקי' הנותרי' בנ"ה צרפם יחד ויהיו ע' דהיינו דינר שלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תן אותו דינר עם הל"ט ויהיו ארבעי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נמצא שנ"ה אמות וט"ו אמות באות עבור מ' דינרי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
צרוף נ"ה וט"ו ויהיה ע' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נמצא שעכשיו אתה יודע ומבין שחשבת כדין וכשורה וד"ל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
[106]ערך אחר אם ישאלך אדם הנה קניתי ק' חבית בעד צ' ליטרין כמה מגיע לחבית אחת | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אז תכתוב תחילה הק' חבית ואח"כ דמיהן דהיינו הצ' ליטרין ותחת הצ' ליטרין תכתוב החבית האחד כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותכפול א' התחתונה על הצ' שלמעלה הימנו א' פעמי' צ' יעלה תשעים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואח"כ תחלק אותה צ' לק"ק פי' מנה כמה פעמי' ק' יש בצ' ולא תשיג ידך אפי' פעם אחת ק' אדרבה חסר לך י' וזה הערך המגיע לחבית א' צ' חלקי' שק' מהן עולין לטרא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
זה מצא מהר"ר וייזיל בספר אחר וכך לשונו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולפי דעתי שאין צריך לטעם זה כי אין מחשבתו של אדם לחילוק צ' חלקי' שק' מהן חלק אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה הטעם ראוי יותר בעיני גם חשבונ' של גוים נמי כזה שאכתוב הנה שאלת ק' חבית בעד ק' חבית בעד צ' ליטרי' כמה מגיע לאחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כתוב כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חשוב בדעתך כמה פשי' יש בצ' ליטרי' אם הין אחד כ' פשי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חשוב ב' פעמי' ב' ויעלה ח' מאות ואלף כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חשוב בשער החילוק כמה פעמי' מאה יש בח' מאות ואלף ותמצא י"ח פעמי' מכוון כך היא החשבון י"ח פשי' לאחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם ישארו לך חלקי' בקטני' אמור כך וכך חלקי' שכך וכך עלו לחלק שלם פי' לטרא ודו"ק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| We wish to evaluate: | ערך אחר הנה בקשנו להעריך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם ישאל אדם קניתי י"ט אמות בגד עבור י"ג דינרי' כמה מגיע לי"ג אמות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אז תעריך כך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כתוב תחילה י"ט אמות ואח"כ דמיו דהיינו י"ג דינרי' ואח"כ י"ג אמות כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותכפול י"ג של מעלה על י"ג שלמטה פי' מנה כמה עולה י"ג פעמי' ותמצא שיעלה קס"ט כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואז תחלק קס"ט לי"ט י"ט פי' מנה כמה פעמי' י"ט בקס"ט ותמצא שיש בו שיש בו ח' פעמי' י"ט ונשארו י"ז חלקי' שי"ט מהן עולה חלק אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה הערך המגיע לי"ג אמות כשקוני' י"ט אמות עבור י"ג דינרי' ח' דנרים וי"ז חלקי' קטני' שי"ז מהן עולין חלק אחד דהיינו דינר אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Likewise, if you wish to evaluate a large amount of merchandise through a small amount of merchandise, I will teach how: | וכן אם תרצה להעריך מקח גדול אחד ממקח קטן אשכילך היאך תעשה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם ישאל השואל הנה קניתי כ"א אמות בגד עבור מ"ג דינרי' כמה מגיע לס"ה אמות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כתוב בתחילה הכ"א אמות ואח"כ המ"ג דינרי' ואח"כ הס"ה אמות [107]אשר בקשת לחשוב כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותכפול ה"ו על ג"ד פי' כמה עולה ג"ד פעמי' ה"ו ויעלה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואח"כ חלוק הטז"ב בשער החילוק כמה פעמי' שיש בהן א"ב ותמצא ג'ג'א' פעמי' ועוד נשארו ב' חלקי' קטני' שא"ב מהן עושי' חלק אחד דהיינו דינר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נמצא אם קנית א"ב אמות עבור ג"ד דנרי' מגיע לה"ו אמות ג'ג'א' דינרי' וב' חלקי' שא"ב מהן דינר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תרצה לידע אם אמת חשבת אם לאו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הבט וראה כמה ה"ו עולה יותר מא"ב דהיינו ד"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
השליכהו בשער הכפל על ג"ד פי' ג"ד פעמי' ד"ד דהיינו בטח"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואח"כ חלקיהו בשער החילוק כמה פעמי' יש בהן א"ב ותמצא 0"ט פעמי' ועוד נשארו ב' חלקי' קטני' שא"ב מהן חלק אחד שלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חבר 0"ט וב' חלקי' קטני' על ג"ד דהיינו הדינרי' השייכי' לא"ב זהו ויהיה גג"א וב' חלקי' קטני' כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והיינו כוונת החשבון ולמבין די | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Boiling Problem |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שאלה היוצא משער הערך הזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה לפניך נ' סאין מים ואם אתה ש' אבשל אות' על האור מתבשלין ונחסרי' בכל יום ז' סאין ועתה שואלי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הילך היאך תעשה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תכתוב תחילה הנ' סאין ואח"כ תכתוב הז' סאין הנחסרי' ביום אחד ותחת הז' סאין תכתוב המ"ה סאין הנשארי' כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותכפול מ"ה שלמטה על ז' שלמעלה הימנה פי' חשוב כמה עולה מ"ה פעמי' ז' ויעלה שט"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תחלוק אות' שט"ו לנ' פי' כמה פעמי' יש בשט"ו ותמצא בו ו' פעמי' נ' ועוד נשארו ט"ו חלקי' קטני' שנ' מהן חלק אחד שלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נמצא כשנ' סאין יחסרו ז' סאין ביום אחד מ"ה סאין יחסרו ו' סאין וט"ו חלקי' קטני' שנ' מהן סיאה שלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שאלה אחרת | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם ישאל השואל הנה יש צ"א סאין מים ואם מתבשלין על האור יחסרו ביום אחד ט' סאין ואם נוסיף עליהם עוד י"א סאין שיהיה הכל ק"ב סאי' ואנו מרבין את האש לפי זה שאם לא היינו מרבי' את האש אז לא היו המים מתמעטי' וחסירי' לפי החשבון הזה כי האור אינו שולט כל כך כשהמים הרבה כמו שאם היו המים מועטי' לפיכך כשמרבי' המים אז [108]צריכי' להרבות האש או אם מתמעטי' אז צריכי' למעט האש נחזור לשאלה ששאלנו כמה יחסרו ק"ב סאין ביו' אחד כשצ"א חסירי' ט' סאין | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הילך היאך תעשה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תכתוב תחילה הצ"א ואח"כ הט' ואח"כ הק"ב כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותכפול ק"ב שלמט' על ט' שלמעלה פי' כמה עולה ט' פעמי' ק"ב ויעלה תתקי"ח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תחלוק תתקי"ח לצ"א פי' כמה פעמי' צ"א יש בתתקי"ח והיינו י' פעמי' צ"א וח' חלקי' קטנים שצ"א מהן חלק שלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נמצא כשצ"א חסירי' ביום אחד ט' סאין יחסרו ק"ב י' סאין וח' חלקי' שצ"א מהן עולי' חקל שלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The checking procedure is as written above regarding the cloth. | והמאזנים על זה כמו שכתבתי לעיל עם הבג' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| This is the rule: the [boiling] water problems are similar to the cloth [pricing] problems, therefore their checking procedure and solving method are similar. | זה הכלל זה השאלות עם המים אות באות כמו השאלו' עם הבגד לכן יש להם מאזני צדק אחד וחשבון אחד ול"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Find a Quantity Problems - Whole from Parts |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Many sciences and problems are derived from this chapter that cannot be counted. | והרבה חכמות ושאלות יוצאין משער זה אשר לא יוכל הסופר לכתוב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Now, I will explain a few of them with God's help. | ועתה אפרש קצת ממנה בע"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| I start by saying: | [ובזה אתחיל ואומר][109] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Tree | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
א הנה לך אילן שחציו בארץ ושלישיתו במים ומעל המים הוא ה' אמות כמה ארכו של כל האילן | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הילך היאך תעשה ותבין העניין | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חשוב כל כך איזה חשבון הקרוב שיכולין לחלק לשני חלקי' ולג' חלקי' זה ו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הסר מן ו' החציה וישאר ג' הסר ג"כ השלישי וישאר א' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אותה א' תכתוב תחילה ואחריה בצד שמאלית אצל הא' תכתוב ו' ותחת הא' תכתוב ה' אמות כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותכפול ה' שתחת הא' על ו' שעומד אחר הא' בשיטה עליונה פי' מנה כמה עולה ה' פעמי' ו' והעולה הוא ל' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אות' ל' תחלק לא'א' פי' מנה כמה פעמי' א' יש בל' ודבר ידוע הוא לכל שיש בל' ל' פעמי' א' וזהו אורך האילן אשר שאלת אם תצא בארץ ושלישו עומד במים ומעל המים הוא ה' אמות וששאלת כמה ארכו הנה ארכו ל' אמות והדמיון כך כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם אתה ירא שאינו כך אז קח רומח שארכו ל' אמו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הסר ממנו חציו זהו ט"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אח"כ הסר ממנו השליש זהו עשרה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וט"ו הרי כ"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נמצא שאין נשאר כ"א ה' אמות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Another example, in order that the reader will be well taught in it and understand its virtues and profit, and it is the question asked: | דומיון אחר למען ירוץ הקורא בו ויבין מעלתו וטובו והוא שאלה ששואלין | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Lance | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה לך רומח שעומד השליש והרביעי' והחומש בארץ ולמעלה מן הארץ ארכו ז' אמות כמה ארכו של כל הרומח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אז חשוב איזה חשבון שיש בו [110]שליש ורביע וחומש וזהו ס' שיכולי' לחלק לג' ולד' ולה' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והסר מס' שליש וזהו כ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הסר כמו כן הרביע הוא ט"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והסיר ג"כ החומש זהו י"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ס"ה מ"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד נשאר לך מס' י"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אז תכתוב תחלה י"ג שנשארו ואחר הי"ג בצדו רחוק מעט תכתוב הס' ותחת הי"ג תכתוב ז' אמות שהרומח עומד למעלה מן הארץ ויהיה כך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תכפול ז' שלמטה על 0"ו שבשיטה עליונה פי' חשוב ומנה כמה עולה ז"פ 0"ו ויעלה ת"ך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תחלק ת"ך לי"ג י"ג פי' חשוב ומנה כמה י"ג יש בת"ך ותמצא שיש בו ל"ב פעמי' י"ג ועוד נשארו ד' שלא יכולנו לעשות מהם י"ג והם ד' מי"ג בשלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נמצא שהרומח ששאלת כשהשליש והרביע והחומש בארץ ולמעלה מן הארץ אכרו ז' אמות ושאלת כמה אורך של כל הרומח ארכו ל"ב אמות וד' חלקי' שי"ג מהן חלק שלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Wall | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ב שאלה אחרת הנה חומה שיסודה שקוע' בקרקע ה' אמו' ולמעלה מן הארץ היא גבוה החציה והשלישי והשביעי' כמה גובהה של כל החומה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אז חשוב איזה חשבון שיכולין לחלקו לב' ולג' ולז' בקל לפי רגע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה לך דרך היאך תמצא אותו חשבון המתחלק לב' ולג' ולז' תמנה זפ"ג כמה כ"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כ"א פעמי' שנים כמה הם מ"ב הרי שמ"ב מתחלקי' לשנים ולשבעה ולשלשה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
[או כלך לדרך זו והכל אחד ב' פעמים ג' הרי ו' ו' פעמים ו' הרי ל"ו וכבר היו לך ו' צרוף ו' עם ל"ו הרי מ"ב הוא] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
או נלך לדרך זו והכל אחד חשוב בפ"ג הרי ו' ו'פ"ז הרי מ"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נמצא שמ"ב הוא דבר המתחלק הוא לשביעיו' או לתשיעיות ולעשיריו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
[וכן לעולם כשישאלך דבר המתחלק לשביעי ולתשיעי ולעשירי] או לכל דבר המתחלק אז תכפול אותו חלק על האחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כגון אם שאל דבר המתחלק לעשיריו' ולתשיעיו' אז תכפול י' על ט' פי' מנה כמה עולה י' פעמי'' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם ישאלך דבר המתחלק לחמישיות ולרביעיו' או למאות ולאלפי' או לכל דבר המתחלק תכפול זה על זה או לכל דבר שישאל השואל שמתחלק כך וכך תכפול זה על זה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם ישאל השואל דבר המתחלק לג' ד' וה' אז תכפול ג' על ד' והעולה שיעלה תכפול על ה' או איפכא תכפול תחילה ה' על ד' והעולה שיעלה בידך תכפול על ג' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועתה נחזור לשאילתינו דלעיל החומה אשר היא חצייה ושליש ושביעי' גבוה על הקרקע ויסודה שקועה בקרקע ה' אמות כמה גובהה של כל החומה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נמצא ששאלנו על דבר המתחלק [111]לב' ולג' ולז' ב"פ ג' היינו ו' ופ"ז היינו מ"ב נמצא שמ"ב הוא חשבון המתחלק לב' ולג' ולז' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועתה נסיר החציה ממ"ב והוא כ"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נסיר כמו כן השליש ממ"ב זהו יהיה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נסיר כמו כן השביעי' ממ"ב זהו יהיה ו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ס"ה מ"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד נשאר ממ"ב א' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נמצא אם היינו שואלי' גובה החמה שיסודה שקוע אמה אחת וחצי ושלישי' ושביעי' למעלה למעלה מן הקרקע אז יהיה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אבל עכשיו צריכין אנו ללמוד ולהעריך כמה מגיע לה' אמו' פי' שיהיה שקוע ה' אמות בקרקע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן נעשה הערך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נכתוב תחיל' האמה האחת ואח"כ רחוק ממנו מעט נכתוב מ"ב אמות ותחת האמה האח' נכתוב הה' אמות ששאלנו ויהיה כך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נכפול ה' על מ"ב פי' נמנה כמה עולה ה' פעמי' מ"ב והעולה הוא ר"י | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נחלק ר"י לא'א' פי' נמנה כמה פעמי' א' יש בר"י ונמצא בו ר"י פעמים א' והנה גובה החומה ששאלנו והדמיון כך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה לך דרך אחר בקוצר היאך תוכל לידע כמה גובהה של כל החומה כשהיא משוקעת בארץ ה' אמות ולמעלה מן הקרקע היא גבוה החציה ושליש ושביעי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תקח ב' פעמי' ה' הרי י' י' פעמי' ג' הרי ל' פעמי' ז' הרי ר"י | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
או נלך לדרך זו ב' פעמי' ה' הרי י' יפ"ג ג' הרי ל' לפ"ז הרי ר"י | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Joint Purchase Problems - If You Give Me |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ג הא לך שאלה אם אדם אחד אומר לך הנה יש כאן דג אחד למכור והנה ג' ואחד מהן אמר לחבירו אתן כל אשר לי וכל אחד מכם יתן החציה ממה שלו ואז יהיה הדג פרוע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תשובה הדג רוצי' ליתן עבור י"ז פשי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והראשון יש לו ה' פשי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והשני י"א פשי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והשלישי יש לו י"ג פשי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
זהו בשלימי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אבל השברי' כגון אם ישאל השואל כשהדג נקנה עבור ח' פשי' או ט' פשי' או י"ח פשי' או ל' פשי' או מ' פשי' או איזה סכום שירצה לשאול [112]ולאותו סכום ששואל אמר האחד אתן כא"ל ואתם תנו שליש שלכם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה אכתוב לך ב' או ג' דמיונות כדי שתוכל להבין כל כיוצא בזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם הדג נקנה עבור ח"פ ואמר הראשון אתן כא"ל ואתם תנו החציה שלכם והשני אמר תנו הג' שלכם והשלישי אמר תנו הרביעי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
צריך שיהא לראשון ב"פ וששה חלקי' שי"ז מהן עושי' חלק שלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולשני ה"פ וג' חלקי' שי"ז מהן עושי' חלק שלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
[ולשלישי ו' פשיטי' וב' חלקים שי"ז עושין חלק שלם] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועתה אראה לך הדרך שתמצא שכן הוא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה אם היה בשלימי' אז היה לראשון ה"פ כמו שפירשתי לעיל ועתה שהוא בשברי' ועתה כשהדג נקנה בח' פשי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אתה צריך להטותו בשער הכפל חפ"ה זהו מ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואח"כ צריך אתה להטותו בשער החילוק ולחלק כמה פעמי' י"ז יש במ' והנה יש ב"פ וששה עודפי' שלא הגיע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נמצא שיש לראשון ב"פ וששה חלקי' שי"ז מהן חלק שלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועתה נפרש כמה שיש לשיני | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם היה בשליש' כמה היו לו י"א עתה שהוא בשברי' אז תאמר לו כמו שאמרת לראשון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
פי' לראשון אמרת ח' פ"ה כן תאמר לשני ח"פ י"א והעולה פ"ח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואח"כ תחלוק פ"ח לי"ז י"ז פי' כמה פעמי' י"ז יש בפ"ח ו והנה יש בו ה' פעמי' י"ז וג' עודפי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן תעשה לשלישי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותאמר אם היה בשלימי' היו לו י"ג פשי' ועכשיו שהוא בשברי' אז תאמר לו ח' פעמי' י"ג הרי ק"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואח"כ [תחלק] ק"ד לי"ז י"ז [פי'] כמה פעמי' י"ז יש בק"ד ותמצא בו ו' פעמי' שי"ז [ב'] מהן עודפי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נמצא שיש לשלישי ו"פ וב' חלקי' שי"ז מהן חלק שלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם השאלה מדג הנקנה בל' פשי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אז תאמר לראשון ל' פעמי' והעולה כתוב ואח"כ תחלקיהו כמו שציויתיך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן לשני וכן לשלשי כדלעיל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועתה כתבת לעיל שיש לראשון ה' ולשני י"א ולשלישי י"ג בשלימי' הנה אראך הדרך שתמצא [113]שהכלל כך הוא של כולם יש כ"ט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
קח ג' חשבונות כשתסיר מהן החציי' והשליש והרשביעי' וישאר בידך ג' ואילו הן ד'ד' וחצי ו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תסיר הרביע מן הד' [וישאר ג'] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והשליש מן ד' וחצי [וישאר ג'] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והחצי מן ו' וישאר ג' מכל ג' אחד ואחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה המורה תועי רוח בינה[note 6] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועתה יען כי אין במור' שלימי' כי ד' וחצי אינו שלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפול ד' ויהיה ח' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפול ד' וחצי ויהיה ט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפול | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ס"ה כ"ט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועדיין אין אנו יודעין כמה מגיע לכל אחד לחלקו ועתה אשכילך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הסיר ב' פעמי' י"ב מכ"ט וישאר ה' והוא חלק של ראשון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן הסר ב' פעמי' ט' מכ"ט וישאר י"א והוא של שני | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן הסר בפ"ח מכ"ט וישאר י"ג והוא חלק של שלישי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נמצא כשהראשון אומר אתן כא"ל וכ"א"מ יתן החציה יהיה ס"ה י"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נמצא שהדג נקנה בי"ז פשי' וכן כולם ודוק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן אם הקוני' ד' או ה' עד אין סוף הרוצה לקנות דג קח מניין השוה בכולם פי' או קונין ג' אז קח המניין כשתסיר השליש והרביע וחציי' וישאר ג' כמו שפירשנו או אם הקוני' ד' קח המניין כשתסיר החציי' והשליש והרביעי' והחמישי' וישאר ד' וכן ה' קח המניין כשתסיר חצי של רביעי' חמישי' ששית ונשאר ה' וכן כולם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכשאין המניין בשלימים תכפול אות' שיהא החשבון שלם ואח"כ כעשית החשבון שלם אם הקוני' ג' תסיר ב' פעמי' ואם ד' תסיר ג' פעמי' הכפיל וכן לעולם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| MS P1088, margin: | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם הקוני' ד' והרביעי אומ' אתן כא"ל וכל אחד יתן רק החומש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אז קח ד' מנינים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אחר תסיר ממנו חצי וישאר ד' וזהו ח' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואחר תסיר ממנו שליש וישאר ד' זהו ו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואחר תסיר ממנו רביעי וישאר ד' זהו ה' ושלישי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואחר תסיר ממנו החומש וישאר ד' זהו ה' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וצריך לקח' זה החשבונות ג' פעמים ואז יהא השליש שלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועתה קח ח' ג' פעמי' זהו ד"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
קח ו' ג"פ זהו ח"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
קח ה' ושלי' ג"פ זהו ו"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
קח ה' ג"פ זהו ה"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
סך הכל ג"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועתה הסר ג"פ ד"ב מג"ז וישאר א' נמצא ש[......] ראובן א' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והסר ג"פ ח"א מג"ז וישאר ט"א זה סך שמעון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והסר ו"א ג"פ מג"ז וישאר ה"ב זהו סך לוי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והסר ה"א ג"פ מג"ז וישאר ח"ב זהו סך יודא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הכל ג"ז והדג נקנה בעד ז"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ודוק ותמצא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ד הנה ג' אנשים הולכי' על השוק ראובן שמעון ולוי לקנות דבר והנה ראובן אמר אתן כל אשר לי וכל אחד מכם יתן הג' שבכיסו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועתה אשכילך הדרך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה יש לך ג' אנשי' ראובן שמעון ולוי ראובן שואל שליש ושמעון ששית ולוי תשיעי' נגדו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כתוב ג' אותיו' לפי שאילת' כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וקח חשבון המועט תקח ממנו השלישי' וישאר ג' נגד בני החיד' זהו ד' וחצי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכותבו תחת הג' הד' וחצי כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וקח חשבון המועט שתקח ממנו הששית [114]וישאר ג' כנגד בני החידה וזהו ג' וג' חומשיהו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותכתבהו תחת הו' כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותקח חשבון המועט שכשתיקח התשיעי' וישאר ג' כנגד בני החידה זהו ג' וג' שמניות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותכתבהו ג"כ תחת הט' כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועתה קח חשבון המועט שתמצא בו חשבון שלם נגד אותן חלקי' שברים שיש לך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כגון חצי חמישי' שמיני' עתה קח חשבון שתמצא בו חצי שלם זהו ב' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וקח חשבון שתמצא חמישי' שלם זהו ה' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וג"כ קח חשבון המועט שתמצא בו שמיני' שלם זהו ח' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תכפול ב' על ה' ויהיה עשרה כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
בזה תמצא שניהם חצי שלם וחמישי' שלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עתה תכפול ותאמר יפ"ד דהיינו חצי ח' זהו מ' כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
בזה תמצא חצי שלם חמישי' שלם שמיני' שלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ובדו' לפכול ה' עם העשר' נגד חלקי שברי' דהן ג' שמי' ואז היה עולה כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אך היית מצוא יותר מצרכך כי כמה תמצא חשבון כמו ב"פ | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולעולם קח חשבון המועט תפשת מרובה לא תפשת תספת מועט תפסת[note 7] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועתה כפול המ' על שכתוב תחת כל אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה מצאת תחת ראובן ד' וחצי כפול 0'ד' על ד' וחצי ויעלה כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכפול 0"ד על שכתוב תחת שמעון דהיינו ג' וג' חומשי' ויעלה כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכפול 0'ד' על שכתוב תחת לוי דהיינו ג' וג' שמיניו' ויעלה כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עת' חבר אילו ג' חשבונות יחד ויעלה כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וזהו המורה את החידה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפול 0ח"א ב' פעמי' ויעלה 0ו"ג ונכיהו מן המורה את החידה שהוא טה"ד ויוותר לך ט"ט כך מעות יש לראובן | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואח"כ כפול ב"פ דד"א והוא הייה כפול לשמעון ויעלה חח"ב נכיהו מן המורה ויוותר לך אז"א כך מעות יש לשמעון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואח"כ כפול ב"פ הג"א שהוא כפול לוי ויעלה 0ז"ב נכיהו מן המורה ויוותר לך טח"א כך מעות יש ללוי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עתה מצאת הכל לראובן ט'ט' ולשמעון אז"א וללוי טח"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והמבין יבין | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Find a Quantity Problems - Whole from Parts |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Tree | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ה אילן התקוע בארץ שלישיתו חמישיתו ותשיעיתו ונותר למעלה מן הארץ י' אמות כמה הית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והא לך דמות אשר תעריך כתוב כזה דהיינו נגד החלקי' ששאלת | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עתה שים דעתך [115]למצא חשבון המועט שנמצא בו החלקי' ששאלת שליש שלם חמישי' שלם תשיעי' שלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכה תאמר ג"פ ה' היינו ה"א ט' פעמי' ה"א יעלה הג"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואינך צריך כ"כ כי תמצאנה בה"ד שהו שליש מן הג"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
קח המועט ולא המרובה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תחסור מן ה"ד כל החלקי' ששאלת דהיינו שלישי' חמישית תשיעי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שליש ה"ד ה"א חמישי' ט' תשיעי' ה' תסרם מן המורה שהיה ה"ד ונותר ו"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עתה כפול האמות הנותרי' למעלה מן הארץ והם 0"א על המורה שהיה ה"ד ויעלה 0ה"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עתה [חלוק ממנו][116] ו"א הנותרי' [בשער החילוק פי' כמה פעמים יש ו"א ב0'ה'ד'] ויעלה החילוק ח"ב וב' חלקי' שו"א מהן עושי' חלק שלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן כל האילן ח"ב אמו' וב' חלקי' שו"א מהן עושין חלק שלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Lance | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ו' הנה רומח לפניך ובא אחד ותוקעו בארץ חציו של כל הרומח ובא אחד והגביה שלישיתו של כל הרומח ובא אחד ותוקעו רביעיתו של כל הרומח ובא אחד והגביה שישיתו של כל הרומח ולאחר מן הארץ הגבהה וזה הגביה הנמוך נותר למעלה מן הארץ א'א' אמות כמה אמות ארכו של כל הרומח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה לך הדרך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כתוב נגד החלקי' ששאלת נגד החצי ב' נגד השליש ג' נגד רביעי' ד' נגד הששית ו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכתוב עליו א'א' אמות הנותרי' כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וקח החשבון המועט שתמצא בו אילו שלם דהיינו ב"א וזה המורה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הסיר מב"א החציה וישאר ו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הוסיף השליש מב"א על הו' [דהיינו ד'] ויהיה 0"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הסיר הרביע מב"א [דהיינו ג'] מ0"א וישאר ז' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הוסיף הששית [של][117] ב"א [דהיינו ב'] על ז' ויהיה ט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אות' [ט'] כתוב בצד אחר ויהיו עיניך רואות את פני מוריך[note 8] שהוא ב"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכפול עליו הא"א הנותרי' למעלה מן הארץ ויעלה כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תחלוק ממנו [בשער החילוק] מה שנותר לך מן המורה שלאחר שחסרת והוסף כל החלקי' זהו ט' כמה פעמים ט' יש בבג"א זהו ד"א פעמי' ונותרי' ו' שאינ' מגיעי' לכלל ט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן אורך הרומח ד"א אמו' ו' חלקי' שט' מהן עולין חלק שלם דהיינו אמה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| This is the rule - when you say: add to it one-third, or one-fifth, or one-quarter, or as much as you want and it is so and so, how much was it originally?
|
זה הכלל כשתאמר שים שליש עליו או חומש או רביע או כל מה שתרצה ויהיה כך וכך כמה היה בתחילה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| I will show you the way to proceed: | אראה לך הדרך אשר תלך בה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Always subtract from the specified number a fraction [whose denominator is smaller by one than] what you intended to add to the unknown number.
|
[118]לעולם הסר מן החשבון המפורשי' חלק אחד יותר מחשבון הנעלם שדעתך לצרף עליו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כגון אם תאמר עשה שליש עליו ויהיה ה' זהו המפורש כמה היה בתחילה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אז הסר מן | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכשתעשה עליו שליש יהיה ה' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
או אם תאמר עשה שביעי' עליו ויהיה ג' זהו ג' המפורש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אז הסר מג' המפור' שמי' ומה שנשאר הוא הסכום מה שהיה בתחילה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואת"ל אם אמת חשבת צרוף שביעי' על הנשאר ויהיה ג' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| And so on do as I showed you. | וכן לעולם כשאר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| But, if you wish to say: subtract one-third and so and so remains, or subtract as much as you want and so and so remains, how much was it originally?
|
אבל אם ת"ל כשתקח שליש ממנו וישאר כך וכך או תיקח ממנו כל מה שתרצה וישאר כך וכך כמה שהיה בתחילה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Divide the remaining number by a [denominator] smaller by one than that of the fraction you subtract, then add the fraction to the remainder, and you will find how much it was originally.
|
אז תחלק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Well | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ז' שאלה הנה לך בור שמימיו נכנסי' בו בג' קני' ויוצאי' ממנו דרך ב' קני' ומהאח' מג' קנים שמימיו נכנסי' בו היה הבור מתמלא מששית היום אם לא היו יוצאין מן הבור מים כלל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תשובה בעשירי' היום מתמלא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואשכילך הדרך כיצד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
קנה האחד שממלא הבור בששי' היום יכול למלא ששה בורות ביו' אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והשני שממלא הבור בחמישי' היום יכול למלא חמשה בורות ביום אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והשלישי שמתמלא הבור ברביעי' היום יכול למלא ד' בורו' ביום אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הרי ט"ו בורות שג' קני' הללו ממלאי' ביום אחד אם [119]לא היו אלו ב' קני' מריקי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ובכמה הן מריקי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
האחד המריק' בחצי היום ממריקי' ב' ליום אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והשני שממריקי' בשלישי' היום ממריק ג' ליום אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הרי ה' בורות שאילו ב' קני' ממריקי' ביום אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועדיין נשארו י' בורות שהג' ממלאי' ביום אחד כשהב' מריקי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נמצא שהבור מתמלא בעשירי' היום | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ובזה תוכל להבין כל כזה וכיוצא בזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Barrel | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כגון ח' אם ישאל השואל יש כאן חבית של ג' ברזות שהוא זב ממנו דרך ברזא האחד זב היא ביום שלם ודרך ברזא השני' זב היא בחצי היום ודרך ברזא השלישי היא בשליש יום וכשהן זבין כולן כאחד בכמה החבית יוצא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תשובה שבשישי' היום יוצא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
היאך הברזא שהוא זב ממנו ביום שלם מריק' חבית אחת ביום אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והשני שהוא זב בחצי יום מריקה ב' חביות ביום אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והשלישי שהוא מריקה בשליש היום מריק' ג' חביות [ביום אחד] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ס"ה כשמריקי' יחד מריקי' ו' חביות ביום אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נמצא שמתרוקן בשישית היום | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ט' אם ישאל השואל הנה בחבית ג' ברזות האחד כשזב לבדו מריקו בשעה והשני לבד ממריקו בב' שעו' והשלישי מריקו בג' שעות וכשיזובו כולם כאחד בכמה מריק' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אבקש לך דמיונות [בחבית המחזיק][120] י"ב הינין שממורק בחצי שעה ובחלק א"א שבחצי שעה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכה תעשה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור בתחילה הברזא שממריק בשעה כמה ממריק בחצי שעה ו' הינין | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והממריק בב' שעות כמה ממריק בחצי שעה ג' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והממריק בג' שעות ממריק בחצי שעה ב' הינין | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נמצא שג' ברזות המריקי' א' ב' ג' מריקי' בחבית המחזיק י"ב הינין בחצי י"א היני' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כמה נשאר עוד הין נמצא שאותו הין שאותו ממריק בחלק י"א שבחצי שעה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Note well and you will understand. | ודוק ותבין | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Fountain | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
י' ועוד שאלו ד' נהרות רצים אל מעיין אחד הראשון ממלאו ביום אחד והב' בב' והג' בג' והד' בד' ואם רצו כולם ביחד בכמה מתמלא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עשה על דרך זה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור אחד חצי שליש רביע [121]אנה ימצאון [ר"ל על דרך המועט כדלעיל דהיינו] בי"ב האחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נמצא בי"ב ימי' ימלאו כ"ה מענייות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כיצד הראשון ממלאו' בי"ב ימים י"ב מענייות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
השני בי"ב ימי' ו' מעיינות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
השלישי בי"ב ימי' ד' מעיינות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הרביעי' בי"ב ימי' ג' מעיינות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
א"כ כ"ה מעיינות בי"ב ימי' מעיין אחד בכמה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אז כפול א' בי"ב ויעלה י"ב חלקי' בשער החילוק [בכ"ה פי' כמה פעמים כ"ה יש בי"ב כנ"ל אש'] נמצא שמעיין האחד מתמלא בי"ב חלקי' שכ"ה מהן יום שלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
לידע כמה חלק מים של כל נהרות לראשון כפול י"ב בא' ויעלה י"ב חלקי' [בשער החילוק] בכ"ה נמצא שמימיו י"ב חלקי' מכ"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפול ו' לשנים בא' ויעלה ו' חלקי' [בשער החילוק] בכ"ה הרי ו' חלקי' מכ"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
לשלישי כפול ד' בא' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
לרביעי ג' בא' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ודוק ותבין | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Multiple Quantities Problems - Selling Eggs |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
י"א חוד חידה ג' נשי' מוכרות ביצי' לאחת יש י' ביצים לשנית יש ל' ולשלישי' נ' וכל אחת תתן ביצה בחליש אחד כמו חבירת' ויפדו מעות שוות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תשובה כל אחת תתן ז' בחליש וכל מה שנותר על השביעיות תתן ביצה אחת בעד ג' חלישים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם היית' ארבע לרביעי' לה ע' ביצים והחשבון כמו כן | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Proportional Division - Inheritance |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
י"ב שאלה אם נפלה ירושה לפני ג' אחים הראשון לקח כל מה שירצה וכן השני וכן השלישי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תשובה הכל היה כ"ד הראשו' לקח י"ג והשני ז' והשלישי ד' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והילך המורה את החידה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם הם רק ג' אז קח לאחרון ג' ואחד יותר הרי זהו ד' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולאמצעי קח כפלים כמו לאחרון וזהו ח' רק אחת תסיר ויהיה ז' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולראשון קח כפלים כמו לאמצעי דהיינו י"ד רק אחת תסיר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן לעולם כמה הן אז קח לאחרון כמניין כולם וא' יותר ואח"כ כפול המבוקש ותסיר אחת | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן אם הם ד' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
קח לאחרון ד' וא' יותר וזהו ה' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולשלישי י' ואחד תסיר זהו ט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
לשני י"ח ואחד תסיר זהו י"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
לראשון ל"ד ואחד תסיר זהו ל"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
בין תבין את אשר לפניך[note 9] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Payment Problem - Digging a Hole |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
[122]יג[123] שוכר את הפועל לחפור בור ארוכה י"ז אמות ברוחב ז' אמות בעד ל"ג זהו' והפועל חפר ג' אמו' במשך י"ז כמה שכרו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תשובה כפול בתחילה בשער הכפל ז"פ י"ז ויעלה טא"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נמצא ששכר את הפועל לחפור קי"ט אמו' ברוחב אמה בעד ל"ג זהו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והפועל חפר ג' אמות במשך י"ז כפול ג' בי"ז ויעלה א"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נמצא שלא חפר רק נ"א אמות והיה לו לחפור קי"ט אמות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואח"כ כתוב קי"ט אמו' ולמטה הימנו כתוב נ"א אמו' כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכפול בשער הכפל א"ה על ג'ג' ויעלה תרפ"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
[חלוק ג"חו"א] לקי"ט פי' כמה פעמי' קי"ט איתא בתרפ"ג ותמצא י"ד פעמי' קי"ט דהיינו טא"א וי"ז חלקי' שלא הגיעו לקי"ט שהוא חלק שלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נמצא שכר הפועל י"ד זהו' וי"ז החלקי' שקי"ט מהן חלק זהוב שלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Find a Quantity Problem - Stolen Purse |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
יד אדם הפקיד לחבירו כיסו מלא מעות ונגנב בפשיעה והמפקיד והנפקד אינם יודעי' כמה היו המעות אשר בו אלא המפקיד זוכר כשמנה המעות בב"ב נשאר א' בג"ג נשאר א' בד'ד' נשאר א' בה"ה נשאר א' בו'ו' נשאר א' בז' יצא מכוון [והנה חוד כמה היו המעות הללו] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תשובה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה אשכילך הדרך היאך תעשה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כתוב כנגד כל מניין השאלה מספר המעוט שתצא בו השאלה פי' שאלת מניין הנמנה בב'ב' וישאר א' נגד [.] זה כתוב ב' ושאלת מניין הנמנה ג'ג' נגד זה כתוב ג' וששאלת מניין הנמנה ד'ד' נגד זה כתוב ד' וששאלת מניין הנמנה ה'ה' נגד זה כתוב ה' ושאלת מנין הנמנה ו'ו' נגד זה כתוב ו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואח"כ כפול ב' על ג' ויעלה ו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפול ו' על ד' ויעלה ד'ב' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפול ד'ב' על ה' ויעלה 0ב"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפול 0ב"א על ו' ויעלה 0ב"ז פי' עשרי' וז' מאות זהו מספר המתחלק לב' ולג' ולד' ולה' ולו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ובהוסיפך עוד אחד שיהיה א"ב אז כשתחלקהו לבגדה"ו ישאר בכל המניין אחת וכשתחלקהו לז'ז' יצא [.] מכוון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן אם ישאל השואל מספר המתחלק לבגדהוז"ח וישאר א' ובט' יצא מכוון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אזי כפול ז"ח ויעלה ו"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואח"כ כפול ו"ה על 0ב"ז והעולה הוא המתחלק לבגדהוז"ח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
[124]ובהוסיפך אחת עליו ישאר א' בכל המניין וב' יוצא מכוון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם ישאלך מניין הנותרי' ג' בכל המנייני' אזי תוסיף ג' על העולה אחר כופלך מספרי' המועטי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן אם ישאלך [מניין הנותר] ד' אזי תוסיף ד' וכן לעולם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Find a Quantity Problem - How Much Problem - Amount of Money |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ט"ו שאלה ממון הוספנו עליו מחציתו שלישיתו רביעיתו חמישיתו שישיתו והכל הוא מ' כמה היה הממון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
בתחילה אשכילך את אשר בלבבי קח מספר המועט המתחלק לחצאין לשליש ולרביע ולחומשי' ולששין וזהו 0"ו פי' ששים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותוסיף על ששים כל החלקי' ששאלת החצי הוא ל' והשליש הוא עשרי' והרביעי הוא ט"ו והחמשי' י"ב והששית י' וצרפם הכל יחד ויהיה פ"ז חבור פ"ז עם ששים ויעלה קמ"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נמצא אם היו שואלי' אותך ממון שהוספת עליו מחציתו שלישיתו ורביעיתו חמשיתו ששיתו ובין הכל קמ"ז כמה תחילת הממון היינו משיבי' תחילתו ששים ועתה שהוא בין הכל רק א' כמה היה תחילתו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אז כתוב אותן קמ"ז בטור העליון ורחוק ממנו קצת כתוב הששים ותחת הס' כתוב המ' שאנו שואלי' ויהיה כך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפול 0"ד ב 0"ו שעליהם ויעלה 00ד"ב פי' כ"ד מאות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תחלק כמה פעמי' זד"א בכ"ד מאות ותמצא שיש בו י"ו פעמי' זד"א ונותר מ"ח חלקי' (חלקי') קטני' שאינן מגיעי' לזד"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נמצא שתחילת הממון י"ו שלמי' ומ"ח חלקי' שזד"א עושי' חלק שלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תחפוץ לשקול אם חשבונך מכוון אז כפול זד"א על י"ו פי' כמה י"ו פעמי' זד"א ויעלה בהג"ב פי' ב' אלפי' וג' מאות ונ"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חבר עמהם מ"ח הנותרי' למעלה שלא עלו לזד"א ויהיה 00ד"ב פי' כ"ד מאות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תוסיף עליהם החציה השלישי' והרביעי' החמישי' והששי' וחבור הכל עם הכ"ד מאות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואח"כ כפול 0"ד פי' מ' פעמי' בזד"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תמצא מכוון מה שיעלה לך אחר שתכפול מ' בקמ"ז כמו החשבון שמצאת [125]בהוסיפך אבגדה"ו על כ"ד מאות אז חשבונך מכוון ואם לאו ודאי
טעית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והבמין יבין | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Divide a Quantity - Loans Repayment |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
י"ו אם ישאלך השואל הנה ראובן שמעון הלוי' שני חובות ראובן הלויה ל"ז זהו' ועלה על כל זהב פשי' לשבוע ועמדו נ"ה שבועו' ושמעו' הלוה לאותו חייב כ"ג זהו' ועמדו ל"ג זהו' ואח"כ בא הבעל חוב ואמ' להן אתן לכם ג' זהו' מן הכל ואתם התפשרו יחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואשכילך הדרך אשר תמצא בו כמה מגיע לראובן וכמה מגיע לשמעון מן הי"ג זהו' כאשר קבלתי מרבי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חשבון כמה רבותו של ראובן שהוא ל"ז על נ"ה ויעלה הג0"ב פשי' פי' ב' אלפי' ול"ה זה היה ריבית שהיה שייך מדינה לראובן | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואח"כ כפול הכ"ג זהו' של שמעון בל"ג שבועות ויעלה טהג"ז שהיה ראוי לשמעון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואח"כ חבר טה"ז עם הג0"ב ויעלה דט0"ב וזהו המורה את החידה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אח"כ כפול הי"ג זהו' על הג0"ב שהיה רבי' שהיה שייך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אח"כ נכהו מן המורה שהוא דטז"ב פי' כמה פעמי' דטז"ב ב' ההדו"ב ותמצא שיש בו ט' פעמי' ונותרי' ט' וג' מאות ואלף שלא יעלו למניין דטז"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נמצא של ראובן שייך מן הי"ג זהו' ט' זהו' ואלף וש"ט חלקי' שלא הגיעו לכלל דטז"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תרצה לידע כמה מגיע לשמעון על הדרך שאמרנו על ראובן אז כפול י"ג בטה"ז שהוא רביתו של שמעון ויעלה זוח"ט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נכיהו מן המורה שהוא דטז"ב פי' כמה פעמי' דטז"ב בזוח"ט ותמצא שיש בו ג' פעמי' ונותרי' אלף ותפ"ה חלקי' שדטז"ב מהן עושין חלק שלם דהיינו זהב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נמצא ששייך לשמעון ג' זהו' והחד"א חלקי' שדטז"ב מהן זהב שלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חבר החד"א עם אלף וש"ט הנותרי' בראובן ויעלה דטז"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תחפוץ לידע חשבון בצימצום אז חלק הי"ג זהו' לפשיטי' [126]ואח"כ כפול בהן ההדו"ב ריביתו של ראובן או דטז"ב רביתו של שמעון ואז יתחלק דטז"ב לחלקי' פשוט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ודוק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Multiple Quantities - Weights |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
י"ז אם ישאלך אדם איך תמצא ארבע משקולות שמשקל כולן רק ארבעי' ואת' תוכל לשקול בהן הן רב הן מעט עד ארבעי' כמה משקל כל אחד בפני עצמו או אם ה' משקל שמשקלם קכ"א ואתה תוכל לשקול בהן הן רב הן מעט כמה משקל כל אחד בפני עצמו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כה תעשה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם תחפוץ להוסיף משקלות הראשון משקלו לטרא השני משקלו ג' לטרין השלישי משקלו ט' לטרי' החמשי פ"א ליטרין הרביעי כ"ז לטרין | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וככה תעשה כתוב בתחילה א' משקלו של משקל הראשון ואח"כ כתוב כך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חברם יחד ויהיה ב' תוסיף עוד א' עליה ויהיה ג' זהו משקל השני | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אח"כ כתוב כך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חברם יחד ויהיה ח' תוסיף עוד אחת ויהיה ט' זהו משקל השלישי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אח"כ כתוב כך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חברם יחד ויהיה כ"ו תוסיף עוד אחת ויהיה כ"ז זהו משקל רביעי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אח"כ כתוב כך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חברם יחד ויהיה פ' תוסיף עוד אחת ויהיה פ"א זהו משקל חמישי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ס"ה קכ"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועל דרך זה תוכל להוסיף | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
או נלך אצל דרך זה למשקל הראשו' קח את אחת לשנים גפ"א לשלישי' ג'פג' לרביעי' גפ"ט לחמישי' ג"פ כ"ז וכן לעולם וסימניך ושלישי' על כולו פי' ג"פ משקל הראשון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Divide a Quantity - Money |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
י"ח אם תרצה לחלק ה"פ לשליש ולרביע ולא ישאר מהן כלום | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור תחילה שליש ורביע בכמה ימצאון בי"ב השליש הוא ד' הרביע הוא ג' חברם יחד הרי ז' וזהו המורה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תרצה לידע בכמה מגיע לזה שיש לו השליש שהוא ד' כפול ד' בה' פשי' ויעלה כ' תחלק בז' ותמצא בו ב' פ"ז ועודף ו' חלקי' שז' מהן חלק שלם הרי שיש לזה ב' פשי' שלימי' וו' שברי' שז' מהן חלק שלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומי שיש לו הרביעי כפול ג' בה' ויעלה ט"ו תחלק ממנו ו' ויעלה ב' פשי' וחלק אחד שז' פשי' שלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הרי שנחלקו ה' [פשוטים] [127]לג' ולד' ולא נשאר מהן כלום | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן אם בידך י"ב פשי' ותרצה לחלקם לחצאין לשליש ולרביעי ולא ישאר כלום | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור חצי שליש ורביע אָנה ימצאון בי"ב החצי ו' השליש ד' הרביע ג' צרוף יחד הרי י"ג וזהו המורה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אח"כ כפול ו' שהוא החציה בי"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אח"כ כפול השליש שהוא ד' בי"ב ויעלה מ"ח תחלק ממנו י"ג תמצא ג' וט' שברי' מי"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן כפול ג' בי"ב ויעלה ל"ו חלק מהן י"ג ותמצא ב' וי' שברי' מי"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן לעולם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Find a Quantity Problem - Whole from Parts - Fish |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
י"ט הרי יש לפניך דג שמשקל גופו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תשובה מנה מספר המתחלק לגדה"ו וזהו ס' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכשתסיר מס' גדה"ו פי' השליש והרבי' והחומש והשישי' ותצרפם יחד ויעלה נ"ז ועודף ג' זהו המורה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפול י' בס' ויעלה ת"ר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תחלק ממנו ג' ותמצא בו ר' פעמי' ג' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן כל משקלו של דג ששאלנו מאתם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Multiple Quantities - Selling Cloth |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כ' שאלה אדם נתן לג' בניו [ל'] אמות בגד למכור והאחד מכר האמה עבור ד' זהו' והשני עבור ה' והג' עבור ו' זהו' וכולם מביאי' מעות שוות זה כזה חוד כמה מעות להג' מן המקח וכמה אמות מכר כל אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תשובה בתחילה אראך כמה שהיה לזה שנתן אמה עבור ד' זהו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור תחילה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
בס' רביעי' ט"ו חמישי' י"ב ששות י' חברם יחד ויהיה ל"ז זהו המורה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואח"כ כפול הט"ו שהוא הרביעי' בל' כמדת הבגד ותמצא ת"נ | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תחלק ת"ן לל"ז ותמצא בו י"ב פעמי' ל"ז וששה חלקי' של"ז אמה שלימה נמצא שהיה לזה שנתן אמה עבור ד' זהו' י"ב אמות וששה חלקי' של"ז מהן אמה שלימה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תחפוץ לידע כמה מעות גבהו לזה שנתן אמה עבור ד' זהובי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
[128]י"ב אמות היו לו וששה חלקים של"ז מהן אמה שלימה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הרי י"ב ד"פ י"ב עולה מ"ח ומן הו' חלקי' הנותרי' עשה כך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלוק כל זהב בז' תפוחי' ותאמר כך אילו היו הו' חלקי' ל"ז [דהיינו דמיהם של בגד אמה] אז היה דמיהן כ"ח תפוחי' עתה שהם רק ו' כמה הם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אז כפול בכ"ח [ויעלה ח'ו'א'] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וחלוק אותו לל"ז ותמצא בו ד' פעמי' ל"ז [ועשרים חלקים] של"ז עושי' תפוח שלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נמצא שזה גבה מ"ח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
לדעת כמה אמו' מכר זה שנתן אמה עבור ה' זהו' עשה כך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפול החומש משישית דהיינו י"ב במניין אמו' הבגד שהם ל' [ויעלה 0'ו'ג'] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותחלק ממנו ל"ז [שהוא] המורה ותמצא בו ט' אמות וכ"ז חלקי' של"ז מהן עושי' אמה שלמה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תחפוץ לידע כמה מעות גבה ט' אמות היינו מ"ה זהו' וכ"ז חלקי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אילו היה ל"ז היו נמכרי' בעד ל"ה תפ | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עשה מכ"א [.] תפוחי' ג' זהו' וחברם יחד עם מ"ה ויהיה מ"ח ויוותרו לך ד' תפוחי' וך' חלקי' של"ז תפוח שלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נמצא שמעו' הראשונות כשיני | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
לדעת המעו' ואמו[ת][129] המוכר אמה עבור ל' זהו' כפול חלק ששי' מס' בל' מניין האמות [דהיינו ל' ויעלה 0'0'ג'] ומן העולה תחלוק ל"ז ותמצא ח' אמות וד' חלקי' של"ז עושי' חלק שלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולדעת כמה מעותיו ח' פעמי' ו' זהו' היינו מ"ח ד' חלקי' הנותרי' אילו היה ל"ז היו נמכרי' במ"ב תפוחי' עתה שהם רק ד' בכמה כפול ד' במ"ב [ויעלה חו"א] ומן העולה תחלק [ל"ז] זהו' ותמצא ד' תפוחי' וך' חלקי' של"ז תפוח שלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נמצא שאין בין זה לזה [במנין][130] המעות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והראשון מכר י"ב אמו' יותר ו' חלקי' ושני מכר ט' אמו' וכ"ז חלקי' והשלישי מכר ח' אמו' וד' חלקי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| וזהו סימנם | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Partnership Problems - for the Same Time - Three Partners |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כ"א ועוד שאלה ג' חבירי' רצו לתת בחבורה מ' לטרי' זהו' לראשון יש לו זהב ששוה הלטרי' ג' זהו' לשני ששוה ה' זהו' ולשלישי יש לו זהב ששוה הלטר' ח' זהו' כמה יש כל אחד לטרי' שוה בשוה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תשובה עשה מספ' המתחלק לגה"ח כיצד כפול ג' על ה' הרי ט"ו כפול ח' על ט"ו ויעלה ק"כ | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הסר מק"כ | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
לדעת כמה לטרי' צריך לשום זה שזה שוה ג' זהו' קח השליש מק"כ [131]וזהו מ' כפול מ' על מ' מניין הליטרין ומן העולה תחלק ע"ט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן לכולם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
לשני כפול החומש מק"ך במ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
לשלישי' כפול מק"כ | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כמה כבר הארכתי בעניין זה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Multiple Quantities |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Four Coins | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כב הרי שיש לפניך ד' מטבעות ששוים מ' פשי' הראשונה שוה החצי והשתות מן השנייה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עשה כך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הראשונה ששוה החצי והשתו' מן השנייה באיזה מספר נמצאי' חצי ושתות שלמי' בששה החצי מהחצי ג' והשתות א' הרי ד' מן הוי' כאילו אמר הראשונ' ב' שליש מן השני | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ושלישית[132] ששוה [שליש][133] ורביע ושתות מן הראשון אילו החלקי' בכמה נמצאון בי"ב השליש ד' הרביע ג' השתות ב' כלול יחד הרי ט' הוי כאילו אמר השליש ג' רבי' מן הראשונה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
[והד'][134] ששוה ד' חומשי' מן (מן) השני אין צריך ביאור דהא מבואר היטב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
לדעת איך תמצאם החלקי' חלוקי' בפי' הבן איזה חלקי' הזכרנו בשאל' חצי ושתות ובכל מקום שתמצא שתות שליש גם תמצא גם הוזכרנו רביעי' וחומש וכל אילו החלקי' נמצא בששי' נמצאת מטבע שנייה ס' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
החצי והשתות גם ל' וי' כללם יחד הרי מ' זו היא מטבע הראשונה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כמה עודף ס' יותר על מ' כ' זהו' שאמרנו בשאלה והנשאר מן השני עודף על הראשון הוא שני שליש מן השלישי' כי מטבע [שלישי ל'][135] כשתסיר גד"ו פי' שליש רביעי' שישית ממ' תמצא שלישי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
לדעת מטבע רביעי' תחלק לה' חלקי' נמצא ה' פעמי' ו' ד' חומשי' מל' הרי כ"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וזהו סדורן מ' ס' ל' כ"ד צרוף אותם יחד הרי קנ"ד זהו המורה את החידה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
לדעת כמה מטבע ראשונה למלאו' מניין ארבעי' כפול מ' במ' שהוא מניין המעות ומן העולה תחלק קנ"ד ותמצא צורתו כך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
פי' י' פשי' וששי' [חלקים] שקנ"ד פשי' שלם זהו סך מטבע ראשונ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אח"כ כפול ס' במ' ומן העולה תחלק קנ"ד ויהיה צורתו כך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
פי' ט"ו פשי' וצ' חלקי' שקנ"ד פשי' שלם זהו סך מטבע שנייה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אח"כ כפול ל' על מ' וחלקהו בקנ"ד ויהיה צורתו כך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
פי' ז' פשי' קכ"ב חלקי' שקנ"ד פשי' שלם זהו סך מטבע ג' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אח"כ כפול כ"ד במ' ומן העולה תחלק קנ"ד וצורתו כך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
פי' ו' פשי' ל"ו חלקי' שקנ"ד פשי' שלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
צרוף יחד י' וט"ו וז' וו' הרי ל"ח[136] [137]צרוף יחד החלקי' שתחתיהן ויעלו ח' וג' מאות דהיינו ב"פ קנ"ד וזהו ב' פשי' וד"ק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Sons | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כ"ג חידה אדם עשיר המחלק נכסיו על פה ואומר לאחד מבניו טול מכיסי זהב בראש ואח"כ טול המעשר מן הנותרי' לשני אמר טול אתה ב' זהו' ועישור נכסי' מן הנותרי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תשובה אמור לפי שהחשבון השבור שבזה הוא עשירי תפחת א' מעשר' ישארו ט' הרי שט' היו הבני' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור אחר זה ט'פ"ט ויעלה פ"א הרי שפ"א היו הזהובי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולכל אחד הגיע ט' זהו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן לעולם היאך שהיה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Motion Problem - Pursuit |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כ"ד אדם שמהלך בכל יו' י' מילין וחבירו מהלך ביום ראשון מיל יום שני ב' מילין וכן בכל ימים מוסיף והולך מיל בכמה ימים מגיע למהלך י' מיל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תשובה חבר י' עם י' ויהיה כ' הסר ממנו אחת וישאר י"ט הרי שבי"ט ימים מגיע [לחבירו] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן לעולם תכפול מהלכו של המהלך קביעיתו והסר אחת ובאילו ימי' הגיעו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם השני מהלך אגה"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תחוסר מן המהלך י' וישיגו בי' ימי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם הוא מהלך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ישיגו [בט'] ימים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והא לך סימן לעולם על המהלך אב"ג שתדע בקיצור כמה מילין הלך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Ordering Problem - Stock of Coins |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כ"ה אז אם ישאלך אדם הנה צבור מעות מונחי' כאן וכולם הונחו על דרך אבגד"הו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תבין בסוף השיטה הזאת ח' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אז קח אמצעי' אותה שיט' שהוא חמשה ואמור ה' פעמי' ט' הרי מ"ה וכך המעות מ"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם סוף השיט' [י'] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אז קח אחת מאמצעי' השיטה ואמור ה"פ י' הרי נ' ותוסיף עוד חצי שיטה דהיינו [ה'] היינו הכל נ"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נקוט האי כללא בידך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כשסוף השיטה בזוגות אז כפול אמצע השיטה על שיטה שלימה ותוסיף חצי שיטה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם סוף השיטה בפרודות כגו' ז' ט' י"א י"ג ט"ו אז כפול אמצעי' השיטה על כל השיטה ואינך צריך להוסיף | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Proportional Division - Three Men Sharing Food |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
[138]כ"ו חידה שנים הסיבו לאכול לחם האחד יש לו ג' ככרות ולשני יש לו ב' ככרות ובא עוד שלישי ואכל עמהם ובגמר סעודה הלך לו והניח לפני השנים ה' פשי' חוד איך יחלקו אילו השנים הה' פשי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דע והבן כמה שהגיע לכל אחד מן הלחמניות תצטרך לומר כיכר שלם ושני שלישי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כמה הפסיד בעל ב' ככרות רק שליש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכמה הפסיד בעל ג' ככרות ככר ושליש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נמצא שנתן עבור ה' שלישיות ה' פשי' זה נוטל ארבע פשי' וזה נוטל פשי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
סליק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Guessing Problem - Chosen Number |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| MS München | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| This is not the language of the book, but the language of the late R. Weysil. | אין זה לשון הספר רק לשון מהר"ר ווייזיל ז"ל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| I have found this in another book. | זה מצאתי בספר אחר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
יחשוב החושב איזה סכום שירצה עד ל"ב והנשאל יתן לו חצי מחשבתו פעם ראשון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ויאמר לו אם יש בו חצי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כגון אם היה מחשבתו ה' ונתן זה לו ב' וחצי דהיינו ז' וחצי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכך יאמר לו עשה החצי לאחד שלם ואותו פעם ראשון אשר היה בו מחצה יתן למשמרת | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם לא היה בו חצי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כגון אם היה מחשבתו ד' דהיינו החצי ב' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
מה טוב ומה נעים[note 10] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואח"כ יאמר לו קח עוד חצי על חשבון אשר בידך וישאל לו אם יש בו מחצה אם לא כמו שכתבתי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וככה יתן לו החצי על החשבון אשר בידו חמשה פעמי' וכל פעם אשר עלה בידו מחצה יתן למשמרת | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואח"כ ילך אל זה השורה וכל פעם אשר היה בו מחצה יקח לו אותו מספר אשר כתוב כאן וישלך אותו בל"ב ל"ב ומה שנשאר בידו הוא המספר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כגון אם היה בפעם שנית ובפעם רביעי' ובפעם חמישי' מחצה א"ב קח מה דכתי' אצל פעם שנית דהיינו אצבע והוא ד"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן לכל פעם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
[139]וכן אם ירצה השואל יחשוב חשבון עד קכ"ח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנשאל אמור לו כמה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כגון אם חושב השואל חשבון שבפעם ראשון ובפעם שלישי ובפעם חמישי ובפעם שביעי' היה בו מחצה דהיינו חשבונם לשורה ראשונה ג"ד ולשלישי ו"ח ולחמשי ח"ד ולשביעי ד"ו צרוף יחד ויהיה אד"ב השליכהו בל"ב ל"ב וישאר ז"א דהיינו החשבון הראשון אשר חשב השואל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
סימניך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Multiple Quantities - Men, Women, Children |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חוד חידה הנה באו הנה נפשות לאכול ויש ביניהם אנשים ונשים וטף וסך כולם עשרים ובא להן בעל אושפיזן ואמר להן תנו לי איש אחד מכם ג' פשי' ואשה ב' פשי' ותינוק חצי פשי' וכן עשו ויעלה הסך של מעות כולם עשרים פשי' חוד כמה היו האנשים וכמה הנשים וכמה הטף | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תשוב איש אחד חמשה נשים י"ד תינוקות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ודוק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דרך אחר הנה באו מאה נפשות למלון אחד וישבו יחד לאכול ויהיה ג"כ ביניהם [אנשים][140] [ונשים][141] וטף ובא ג"כ להן בעל אושפיזין ואמר להן תנו לי כל איש עשרה פשי' ואשה ה' פשי' ותינוק חצי פשי' וכן עשו ויעלה הסך של מעות מאה פשי' חוד כמה אנשים וכמה הנשי' וכמה תינוקות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תשובה איש אחד ותשעה נשים ותשעים תינוקו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Double False Position
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Question by R. Israel from Prague: | שאלה מפי הר"ר ישראל מפראג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| A number that we add to it, how much was it originally? | ממון שהוספנו עלינו כמה שהיה בתחלה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כגון אם הוא ג' תוסיף עליו ג' וגם מחציתו ורביעתו ויהיה הכל עשרים או ל' או מ' או כפי רצונך כמה שתרצה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Write two false numbers and use them to calculate the truth, how much it was originally. | ותכתוב שני חשבונות שקרים ותחשוב מהם האמת כמה היה בתחילה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה לך דימיון כגון ממון שהוספנו עליו כמו שהיה בתחילה ומחציתו ורביעתו ויהיה הכל עשרים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אז חשוב אם היה בתחלה המעות ד' זהו' והוספנו עליו עוד ד' ועוד מחציתו שהוא ב' ורביעתו שהוא א' והנה הכל ביחד א'א' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה הוא שקר כי היה ראוי להיות הכל 0"ב כי שאילתינו כשהוספנו עליו כמו שהיה בתחילה ומחציתו ורביעתו ויהיה הכל עשרים וזה אינו רק א'א' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אז חשוב כמה הוא מן א'א' עד 0"ב ויהיה ט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אותו ט' כתוב תחת ד' שאמרנו שהיו המעות בתחילה כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואח"כ חשוב אם היו המעו' בתחילה ח' והוספנו עליו כפי שאילתינו עוד ח' ומחציתו שהוא ד' ורביעיתו שהוא ב' והנה הכל ביחד ב'ב' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
א"כ זהו ג"כ שקר כי הוא ראוי להיות הכל ביחד 0"ב כמו ששאלנו כי שאלנו כשהוספנו עליו כמו שהיה בתחילה ומחציתו ורביעתו ויהיה הכל 0"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אז תנכה 0"ב מן החשבון כפי שאילתנו ויהיה יותר ב' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אותו ב' כתוב תחת ח' שאמרנו שהיה המעות בתחילה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכתוב ח' בצד שמאל של ד' וגם ב' הנשאר מן החשבון כתוב בצד שמאל על ט' כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה צרוף ט"ב ביחד כי הם נשארים מן החשבונות השקרים ויהיה א'א' וזה המורה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אח"כ כפל ב' שתחת על ד' ויהיה ח' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אותו ח' כתוב שלא תשכח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואח"כ כפול ט' שתחת ד' על ח' ויהיה ב"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וקח ח' שכתבת כבר ושים אותו על ב' ויהיה עשרה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כתוב גלגל במקום ב' ומחוק ד' וכתוב במקומה ח' ויהיה 0"ח פי' פ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואח"כ חלוק בשער החילוק כמה פעמים א'א' יש בשמונים כי הוא המורה ותמצא בו ז' פעמים ועוד ג' חלקים שא'א' מהן חלק שלם פי' שלם זהב שלם וכך היא המעות בתחילה ששאלנו והמבין יבין | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The above calculation is correct when one of the two false numbers is deficient and the other is exceeding. | וזה החשבון דלעיל מכוון כששני חשבונות השקרים אחד פחות ואחד יותר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| But, if the two false numbers both exceed the [original] number, or both deficient, I will teach you what to do then: | אבל אם שני חשבונות שקרים כל אחד יותר על חשבון היוצא או שניהם חסרים אשכילך האיך תעשה אז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Subtract the smaller remainder from the greater remainder and what you are left with is the denominator. | תנכה החשבון המועט הנשאר מן חשבון המרובע ומה שישאר לך זהו המורה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| When multiplying the two numbers by each other, subtract also the smaller number from the greater. | וכשכפלת שני חשבונות יחד אז תנכה גם כן חשבון המועט מן המרובה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה אכתוב לך דימיון כגון אם יש לך סך מעות וכפלת עליו סך שהיה כבר ומחציתו ורביעתו ויהיה בין הכל 0"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אשכילך האיך תעשה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם תאמר שהיה סך מעות ח' זהו' והוספנו עליו עוד ח' ומחציתו שהוא ד' ורביעתו שהוא ב' ויהיה [ס]ך הכל ב'ב' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה שקר כי היה ראוי להיות סך הכל 0"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אז תנכה 0"ב וישאר ב' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אותו ב' כתוב שלא [ת]שכח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואז תחשוב חשבון השני ותאמר אם היו סך המעות ו"א והוספנו עליו ו"א ומחציתו שהוא ח' ורביעיתו שהוא ד' ויהיה בין הכל ד"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה שקר גמור כי היה ראוי להיות 0"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אז תנכה 0"ב שהוא סך היוצא מן מעות מן ד"ד וישאר ד"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואח"כ ב' הנשאר מן חשבון הראשון מן ד"ב הנשאר מן חשבון השני וישאר ב"ב וזהו המורה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואז כפול ב' שתחת ח' על ו"א ויהיה ב"ג כתוב שלא תשכח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואח"כ כפול ד'ב' על ח' ויעלה ב'ט'א' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אז תנכה החשבון הראשון אשר כפלת שהוא ב"ג מן החשבון השני שהוא ב'ט'א' כי הוא חשבון המועט וישאר 0'ו'א' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אז חלוק בשער החילוק כמה פעמים ב'ב' יש בו כי הוא המורה ותמצא שיש בו ז' פעמים וגם ו' חלקים שב'ב' מהן חלק שלם דהיינו זהו' והמבין יבין סליק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Additional Word Problems |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Mantova, Comunità Ebraica MS ebr. 8/16 |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| I will reveal to you the chapter on fractions: | והנה אגלה לך שער השברים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם נשאל לך השואל על פת אחת לכמה חלקים שצריך אתה לשבר הפת שתמצא בה שלישית ורביעית וחמישית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אז תכתוב כך השאלה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותאמ' ג' פעמים ד' ויעלה ב"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותכפול פעם אחת הב"א תוך הה' אז יעל' לך 0"ו וזה חלוקים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שתמצא שלישית ורביעית וחמישית דהא 0"ב שלישית מ0"ו ורביעית ה"א וחמישית זהו ב"א סליק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| I will reveal to you the chapter on ratio and this its example: | והנה אגלה לך שער הערך וזהוא דמיונו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם נשאל לך השואל הנה לפניך ב"א חביות שנקנין עבור ו' זהובי' ט' חביות כמה הן עולין | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אז תכתו' כך השאלה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואז תאמר ו' פעמי' ט' ויעלה לך ד"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אז תחלוק הב"א מד"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותאמר כמה א' בה' והא' הוי תוך הה' ה' פעמים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אבל לא תוכל ליקח ה' פעמים דאל"כ לא תוכל ליקח הב' מן הד' ה' פעמים וכמה פעמים שתקח הא' מן הה' כך הרבה פעמים צריך אתה ליקח הב' מן הד' אלא לא תוכל ליקח הא' מן הה' אלא ד' פעמים ואז נשאר לך ד"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והד' תכתו' למעלה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דה"ד הוי הזהו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואז צריך ליקח הב' מן ד"א נמי ד' פעמי' אז נשאר לך ו' וזהו ג' גדולי' שעולי' י"ב על הזהוב אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונמצא לכשתקנה ב"א חביות עבור ו' זהובי' שט' חביות עולים ד' זהובי' וחצי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כן תעשה לכל פעם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| I will explain to you another ratio: | ועוד אפרש לך ערך אחר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שנים שהלוו על חוב אחד ד"ג זהובי' ולאחד יש לו בחוב ה"א זהובי' ולשיני יש לו בחוב ט"א זהובי' ולקחו שנים לרבית ג"א זהובי' כמה יש לכל אחד בה ג"א זהובי' לפי הערך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה אפרש לך בקיצור איך תעשה שתמצא היושר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אז תכתו' כל אחד לחוד וכך תכתו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הריבי' למעלה ואח"כ המעות שיש לו בחוב ואח"כ הסך כולה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה דומיונו שכתו' מבראי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותכפל המעות שלו תוך הריבית ותאמ' פ' א'פ'א' ואח"כ תאמ' א'פ'ה' שהוא ה' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואח"כ תכתו' עוד פעם אחת ה"א תחת הג' שלמעלה ויהיה כך דמיונו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותאמ' גפ"א שהוא ג' ותשים על הה' שלמעלה אז יהיה ח' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותאמ' עוד פ"א גפ"ה שהוא ה"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אז תמצא ה'ט'א' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אז תחליק הד"ג מן הט"א ותאמ' כמה יש ג' בתוך ט"א והוא הוי ביה ו'פ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אבל תוכל לקח ו' פ' כי אז אין נשאר לך אלא ה'א' ולא תוכל ליקח ו'פ'ד' מה"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אלא לא תוכל ליקח אל ה'פ' אז נשאר לך הד' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והה' תכתו' למעלה כמו כן | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותקח ה'פ'ד' מן ה'ד' אז תשאר לך ה"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אז יהיה לזה בחלקו ה' זהוב' והב' גדולי' שעולים ד"ג על זהוב אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועשית' גם כן לצד השני ותמצא שיהיה לשני בחלקו ז' זהובי' וט' גדולי' שעולי' ד"ג על זהוב אחד סליק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Boiling Water Problem | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| I will explain to you another ratio: | הנה אפרש לך ערך אחר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם ישאל לך השואל כי הנה ה"א סאין מים לפניך ואם מתבשלין על האור יום אחד אז הוי מתמעטין ז' סאין הנה אם היו רק ג"א סאין על האש והאש הוי מממועט לפי זה הערך כמה הוי מתמעט מן הג"א סאין ביום אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אז תכתו' כך השאלה כדכתי' מבדאי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותעשה כמו שפרשתי לעיל תכפול הג"א תוך הז' ותמצא א"ט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אז תחלוק ה"א מן הא"ט ותמצא שהוי הג"א סאין מתמעט ביום אחד ו' סאין וחלק אחד שה"א עושין על סאה אחת סליק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The [chapter] on ratio | השורש מערך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שנים שהלוו על חוב ט' זהו' לאחד יש ה' זהו' ולאחד ד' זהו' ולקחו לרבית י"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונמצא דשייך לכל אחד בזהוב י"ג תשיעיו' לרבית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונמצא דשייך לזה שיש לו ה' זהו' ה'פ' י"ג תשיעיו' שהם וו' תשיעיו' וכשתחלוק ממנו ט' שהוא המור' פי' כל ט' תשיעיות הם רבי' זהוב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכך תעשה גם לשני סליק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| I will explain to you a ratio of a ratio: | והנה אפרש לך ערך מתוך ערך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה אחד שלוה על חוב ט' זהובי' והם עומדים ד"א שבועות ואחד שלוה על חוב א"א זהובי' והם עומדי' ה"א שבועות ולקחו שניהם ליחד ריבית ז' זהובי' כמה יש לכל אחד בחלקו לפי הערך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה אפרש לך בקיצור איך שתעשה שתמצ' האמת | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תכתו' לכל אחד לבדו כמה שהכתב מבדאי הריבי' לעי' ואח"כ המעות ואח"כ השובועו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותשים צד אחד לפניך איזי שתרצה ותכפל המעות תוך השבועות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותאמ' ט'פ'א' שהוא ט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואח"כ תאמ' טפ"ד ותמצא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותעשה ג"כ לצד השיני ותמצא הו"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אז תחבר אותם ליחד וב"א והו"א ותמצא אט"ב וזה תרשום לפניך כי הוא המור' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואח"כ תכפול כל אחד ואחד וב"א והו"א תוך הריבית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכשתכפול וב"א תוך הז' אז תמצא בח"ח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותחליק אט"ב הנזכרים לעיל מן בח"ח ותמצ' אט"ב תוך בח"ח ג"פ והג' הוא הזהו' שיהיו לו בחלקו וגם ט' נשאר לך מן החילק הוא הוי ט' גדולי' שעולי' אט"ב על אט"ב על אט"ב זהוב אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותעשה ג"כ לצד השני ותיכפול הוא תוך הז' ותמצא ה"הא"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותחליק ג"כ אט"ב מן ה"הא"א ותמצא אט"ב תוך ה"אא"א ג"פ שהוא הזהו' שיהיו לו בחלקו וגם נשאר לך בח"ב שלא יכולים להשיג לכלל אט"ב שהוא גדולים שעולים אט"ב על זהו' אחד ונמצא שיהיו לזה בחלקו ג' זהו' ובח"ב גדולי' שעולי' אט"ב על זהוב אחד סליק דוק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם נשאל לך על זה העיגול כמה כי יאמר לך החץ וחצי היתור אותו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אז תאמר כמה היתור חצי הוא הד' אמות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אז תאמ' ד'פ'ד' והוא ו"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותאמ' כמה אורך החץ והוא אמה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אז תחלק האמ' מן הו"א ותאמר כמה א' בתוך ו"א והוא ו"א והוא ו"א פ' בתוכה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| ? | אז תעשה אורך החץ שהוא א' על הו"א אז יהיה ז"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וקח הו"א אורכו העיגול | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן תעשה לכל פעם תכפול היתור תחלק החץ מן הנכפל ודוק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה אפרש לך שאלה שנקר' הסולם אם נשאל לך על סולם אחד שהיא גבוה עשרה אמות ואם נישג הסולם למעלה ב' אמות כמה אמות השיג למטה מחומה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אז תעשה כך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תאמ' כמה אורך הסול' אורכה עשרה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותאמ' י'פ'י' שהי' ק' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותאמ' כמה אורך החומה מן הארץ עד ראש הסולם והיא ח' אמות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותאמ' ח'פ'ח' והוא ד"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותחלק הח' מן | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותעשה שורת בהו'ג' והשורת בהוג הוא ו' זה אורך הקרקע מן החומה עד הסולם זליק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
בזה הוכיח דלאכח מה שבריבוע אמה על אמה שהאלכסון שלו אמ' ותרי חומשין | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כי זה 0"א אמות על 0"א אמו' ודוק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Moscow, Russian State Library, Ms. Guenzburg 714/4 |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
[142]שאלה הנה שוורים הרבה בזה אחר זה ובענין זה בכל פעם כשקניתי הראשון בעד סך א' אז קניתי אותו שור של אחריו בעד כפל הראשון וכן השור השלישי קניתי ג"כ בעד כפל שקניתי אותו שלפניו וכן השור הד' וכן השור הה' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כגון הא' קניתיו בעד א' זהוב הב' בב' זהובי' הג' בד' וכן כולם והנה השאלה כמה כל המעות הללו בסך אחת | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תשובה זאת ועוד אחרת דוגמא זה למצוא ולחשוב ולעמוד על בוריו תעשה בענין זה ואופן זה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
בתחלה תראה ותבין כמה הוא סך הראשון שקנית בו השור הראשון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואח"כ תראה ג"כ כמה הוא הסך האחרון ב' פעמים בשער הכפל גדול | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותחסר ממנו בשער החיסור הסך הראשון שקנית בו השור הראשון ומה שישאר בידך כשחסרת ממנו הסך הא' הוא סכום כל המעות שקנית בהם כל השוורים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה לך הדמיון כגון הראשון קנית בעד א' זהוב והשני בעד ב' והשלישי בעד ד' והרביעי בעד ח' והה' בעד י"ו והו' בעד ל"ב והז' בעד ס"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה תראה אתה שהסך האחרון שקנית בו השור הז' היינו השור האחרון הוא ס"ד זהובים א"כ תחשוב בשער הכפול הגדול ב' פעמים ס"ד ויהיה [143]ס"ד ויהיה קכ"ח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אז תחסר מן קכ"ח הסך הראשון שקנית בו השור הא' שהוא א' זהוב וישאר בידך קכ"ז זה כל כך מעות קנית כל השוורים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן כל פעם תחסר מן הסך האחרון סך הראשון אחרי אשר כפלת אותו ב' פעמים ולאו דוקא אם הסך הראשון א' שהוא הדין אם הסך הא' ב' או ג' או ד' או כמה שהוא בתחלה תחסר מן הסך האחרון כשכפלת אותו ב"פ ואל תשנה מעניין זה כי חשבון א' הוא ואמת | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שאלה אם תרצה לידע ולחשוב חשבון מה שתרצה בעניין זה בכל פעם אותו חשבון שלאחריו הוא ד' פעמים כמו אותו חשבון שלפניו כגון א' ד' י"ו ס"ד ס"ד רנ"ו ותרצה לידע ולחשוב כמה הוא סך אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אשכילך בדרך זו תלך למען תצליח ותשכיל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה אראך הדרך אשר תלך בה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
בתחלה תראה ותבין ג"כ על הסך הראשון ועל הסך האחרון כמה הם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמנם אינו צריך לכפול סך האחרון כמו שהראיתיך לעיל . רק קח בידך כמו שתמצא אותו עתה ותחסר ממנו סך הראשון מן סך האחרון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אז תוכל לחלק הנשאר בידך לג' חלקים שוים וזה קל להבין לכל בר משכיל אשר הערה את נפשו למלאכה היקרה הזאת | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כי אמשול לך [144]משל בדמיון קטן ואשכילך בו למען תבין ממנו ותראה בעיניך שתוכל לחלק הנשאר בידך לג' חלקים אחרי שחסרת ממנו הסך הראשון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה לך הדמיון כגון אם תרצה לידע ולחשוב בסך א' א' ד' י"ו ס"ד רנ"ו [...] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תראה עתה שהסך האחרון הוא רנ"ו והסך הא' הוא א' ותרצה לחשוב כל הסכומין בסך אחד אז קח הסך האחרון היינו רנ"ו ותחסר ממנו הסך הראשון היינו א' וישאר לך רנ"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואח"כ תחלק בשער החלוק כמה יש ברנ"ה הג' כי תמצא שיצא החשבון לג' חלקים שוים ולא ישאר לך מהם כלום אחר אשר חסרת ממנו הסך הראשון היינו א' ואם אתה עושה כן תמצא שיש בו פ"ה פעמים ג' ברנ"ה והיינו השליש מן הרנ"ה כי פ"ה פעמים ג' היינו רנ"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם כן תראה עתה שיצא החשבון בג' חלקים שוים אחרי שחסרת ממנו הסך הראשון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אח"כ קח אותו השליש מן רנ"ה דהיינו פ"ה וחבר אותו אל רנ"ו כי כן היה הסך האחרון מתחלה קודם שחסרת ממנו הסך הראשון ותמצא אחר החיבור שיצא לך תר"ל (?) והיינו כל החשבון אשר חפצת לידע כי כשתחבר ביחד א' ד' י"ו ס"ד ס"ד רנ"ו יעלה לך תר"ל (?) | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותן לבך להבין ולא לשכוח בכל [145]פעם לחסר החשבון הראשון מן סך האחרון ויצא אחר החיסור לג' חלקים שוים אח"כ תוכל ליקח החלק הג' היינו השליש ולחברו אל הסך האחרון כמו שהיה בתחלה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולאו דוקא אם הסך הראשון א' הוא הדין אם הסך הראשון ב' או ג' או ד' או ה' או כמה שתרצה תוכל בכל פעם לחסר אותו מן הסך האחרון ויצא לך הסך בכל פעם אחר החיסור לג' חלקים שוים וחבור אותו השליש לסך האחרון כמו שהיה בתחלה וזהו חשבון ודוק תם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Give and Take Problems | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
[146]אדם הלך לשוק וכפל מעותיו והוציא י"ב פשוטים ועוד הלך לשוק אחר וכפל הנשאר בידו והוציא י"ב פשוטים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עשה כך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
בעבור הראשון נצטרך להוציא החצי מן הי"ב שהוציא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ובעבור השני נקח הרביעי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ובעבור השלישי נקח השמינית מי"ב שהוא פשוט וחצי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הסר א' פשוט וחצי מי"ב ישארו י' וחצי וכן היה הקרן שהוליך לשוק הראשון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועל זה הדרך תוכל לעשות ואם ילך לכמה שווקים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כי בעבור השוק הרביעי נסיר מן הי"ב פ' שהיה מוציא בכל פעם חלק א' מי"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ובעבור החמישי נסיר חלק א' מל"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ובעבור השישי נסיר חלק א' מס"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר תרבה השווקים כן תכפול מה שיש בידך ועל הכפול חלק מה שהוציא ומה שיעלה בחילוק הסר ממה שהוציא והוא המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אדם א' הלך לפרדס א' ללקוט תפוחים ויש לו ללקוט מהם כל כך שיתן לשוער הפנימי [147]של הפרדס חצי התפוחים שלקט וא' יותר ולשוער השני מן השער הב' יש לו ליתן החצי מן התפוחים שנשארו בידו וא' יותר וכן יש לו [ליתן] [...] לששה שערים שיש לפרדס דהיינו לתת לכל שוער החצי וא' יותר [...] תפוחים לא פחות ולא יותר עשה כך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תפוש מספר הה' שהוא חייב להביא ותוסיף עליו א' הרי ו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפול ו' ויהא י"ב הרי שער א' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הוסיף עליו א' הרי י"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפול אותם ויהיו כ"ו הרי ב' שערים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תוסיף עליו א' ויהיו כ"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפול אותם ויהיו נ"ד הרי ג' שערים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תוסיף עליהם א' ויהיו נ"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפול אותם ויהיו ק"י הרי ד' שערים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תוסיף עליהם א' ויהיו קי"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפול אותם ויהיו רכ"ב הרי ה' שערים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תוסיף עליהם א' ויהיו רכ"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפול אותם ויהיו תמ"ו הרי ו' שערים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הרי שיש לו ללקט תמ"ו תפוחים בין הכל וישארו בידו ה' תפוחים להביא לא פיחות ולא יותר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם יש לו ללקט מהם כל כך שיתן לשוער הפנימי החצי ממה שלקט וא' יותר ולשוער הב' חצי הנשאר וב' יותר ולשוער הג' חצי הנשאר וג' יותר [148]ולשוער הד' חצי הנשאר וד' יותר וד' תפוחים יש לו להביא לא פחות ולא יותר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עשה כך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תפוס מספר הד' שיש לו להביא הוסף עליהם ד' שיש לו להוסיף לשו[ער] [...] שער א' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הוסף [עליהם ג' וי]היו י"ט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפלם ויהיו ל"ח הרי ב' שערים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הוסף עליהם ב' ויהיו מ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפלם ויהיו פ' הרי ג' שערים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הוסף עליהם א' יהיו פ"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפלם ויהיו קס"ב הרי ד' שערים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והתפוחים שלקט היו קס"ב והנשארים בידו ד' תפוחים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Find a Quantity Problems - Whole from Parts | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חנית מעוכה בארץ שלישיתה ורביעיתה ולמעלה מן הארץ היא פ' אמה כמה אמות היא בין כולה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עשה כך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תמצא מספר שיש לו שליש ורביע והוא י"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ושליש ורביע מי"ב הוא ז' והוא התחוב בארץ | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולמעלה מן הארץ נשאר ה' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם כך אמור כך אם ה' שווה י"ב כמה ישוה כ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפול אותם כ"פ י"ב יעלו ר"מ | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקם על ה' יעלו מ"ח בחלוק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
א"כ אורך החנית מ"ח אמות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ראובן שכר שמעון שיבנה לו בית א' בל' יום והיום שיעבוד בבנין ירויח ג' ליט' והיום שלא יעבוד יפסיד ד' ליט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עשה כך אין ספק כי מה שירויח בד' ימים לחשבון ג' ליט' ליום יפסיד בג' ימים לחשבון ד' ליט' ליום | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חבר ד' עם הג' יהיו ז' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפול ג"פ ל' יהיו צ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקם על [ז' יעלו] [150][י"ב ימים וו' חלקים של ז' והם הימים שלא עבד בה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואחר הכפיל ד"פ ל' ויעלו ק"כ | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקם על ז' ויעלו י"ז יום וחלק א' מז' ביום] וכן ימים עבד בה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם יאמר כי היום שיעבוד בבית ירויח ל"ו פשוט והיום שלא יעבוד יפסיד מ"א פשוטים ובסוף ל' יום לא הרויח ולא הפסיד כלום | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עשה כך אין ספק כי מה שירויח במ"א יום לחשבון ל"ו פשוטים ליום יפסיד בל"ו יום לחשבון מ"א פשוטים ליום | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תחבר ל"ו עם מ"א יעלו ע"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפול ל"ו פעמים ל' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקם על ע"ז ויעלו בחלוק י"ד יום שלם וב' חלקים מע"ז ביום וכך ימים לא עבד בבית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועוד כפול מ"א פעמים ל' ויעלה אלף ור"ל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקם על ע"ז ויעלו בחלוק ט"ו ימים שלמים וע"ה חלקים מע"ז ביום | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Divide a Quantity - Sharing Food | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
[151]ב' אנשים היו יושבין לאכול לאחד היו ב' לחמים ולשני ג' לחמים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כמה חלק מכל לחם אכל כל א' מהם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
א' לחם וב' שלישיות מלחם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
א"כ אותו שהיו לו ב' לחמים ואכל א' לחם וב' שלישיות לא הפסיד כי אם שליש לחם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואותו שהיו לו ג' לחמים ואכל א' לחם וב' שלישיות הפסיד א' לחם ושליש שהם ד' שלישיות לחם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
א"כ אותו שהיו לו ב' לחמים יקח א' פשוט כי הפסיד שליש לחם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואותו שהיו לו ג' לחמים יקח ד' פשוטים כי הפסיד לחם א' ושליש שהם ד' שלישי לחם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Motion Problem - To and From - an Ant Climbing a Tower | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
מגדל שהוא גבוה כ' אמה ונמלה א' רוצה לעלות למעלה ובכל יום עולה שליש אמה ובכל לילה יורדה רביע אמה כמה יתרון יש לה בכל יום ובכמה ימים תעלה למעלה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור תחלה כמה הוא יותר השליש מן הרביע א' חלק מי"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הרי שבי"ב ימים היא עולה א' אמה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ובעבור שהמגדל היא גבוה כ' אמות אמור י"ב פעמים כ' הרי ר"מ הרי שבר"מ ימים היא עולה לראש המגדל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| How Much Problem – Money | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הרי שיש לך מעות והוצאת מהם השליש והרביע [152]והחומש והם ט' פשוטים כמה יהיו הנשארים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור תחלה שליש ורביע וחומש ימצאו בס' השליש הוא כ' הרביע הוא ט"ו החומש הוא י"ב וכללם יהיו מ"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הרי שהשליש והרביע והחומש שהם ט' פשוטים הם מ"ז חלקים מס' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נמצא שהנשאר [...] י"ג חלקים מס' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולכן אמור אם מ"ז חלקים מס' שוים ט' פשוטים י"ג חלקים מס' כמה שוים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור י"ג פעמים ט' הם קי"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקם במ"ז יבואו כ"ג חלקים ממ"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נמצא שהיו בכיס י"א פשוטים וכ"ג חלקים ממ"ז ומה שהוציא הוא ט' ומה שנשאר היו ב' וכ"ג חלקים ממ"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן לכל חשבון שתרצה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אדם א' יש לו קנקן שמחזיק ח' כוסות של יין ורוצה לחלקו לשני אנשים וליתן לכל א' ד' כוסות ואין לו רק שני כלים שהא' מחזיק ג' כוסות וא' ה' היאך יעשה לחלק אותם וליתן ד' לכל א' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תחלה ימלא הכלי שמחזיק ג' כוסות וישים אותם בתוך הכלי המחזיק ה' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואח"כ ימלא פעם אחר הכלי המחזיק ג' ויריק כמו כן באותו הכלי המחזיק ה' הרי שהכלי המחזיק ה' מלא ונשאר בכלי המחזיק ג' כוס א' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אח"כ יריק הכלי מלא של ה' כוסות בתוך הקנקן המחזיק ח' ונמצא [153]שבתוך הקנקן גדול יש בו ז' כוסות ובכלי המחזיק ג' יש בו כוס א' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אח"כ יריק הכלי של ג' כוסות שיש בו כוס א' בתוך הכלי המחזיק ה' ויחזור וימלא הכלי המחזיק ג' מתוך הכלי המחזיק ח' ויריק בתוך הכלי המחזיק ה' ונמצא עכשיו ד' כוסות בתוך הכלי המחזיק ח' וד' כוסות בתוך הכלי המחזיק ה' ועם זה היין חלוק בשוה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Joint Purchase Problem - If You Give Me - Amounts of Money | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שלשה חברים היו ואמר הא' לשני חבריו דעו כי כל מה שבכיסי והחצי משניכם עולה ס' ויען השני ויאמר כל מה שבכיסי ורביע משניכם עולה ס' ויען השלישי כל מה שבכיסי ושליש משניכם עולה ס' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
מצאנו ג' מספרים והם ה' י"א וי"ג שהם כעין השאלה הזאת | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כי ה' אומר לי"א ולי"ג אני וחצי שניכם י"ז וי"א אומר לה' ולי"ג אני ושליש שניכם י"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנך רואה שאלו אמרו מחובר שלשתנו י"ז יהיה לא' ה' ולשני י"א ולשלישי י"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועכשיו שאמרו מחובר שלשתנו יחד ס' נשוב לערכים ונאמר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כערך ה' לי"ז כן ערך הקטן אל ס' הוא ששאל לחבריו החצי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכערך י"א לי"ז כן ערך השני לס' והוא ששאל [154]לחבריו השליש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכערך י"ג לי"ז כן ערך הגדול אל ס' והוא ששאל לחביריו הרביע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן תערוך לכל הסכומים שיאמרו שלשתם תשים המורה ה' וי"א וי"ג ודרך הערכים הוא כענין | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור אם בעת שהמחובר הוא י"ז יהיה חלק הקטן ה' בהיות המחובר ס' כמה הם חלקיו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור ספ"ה הוא ש' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וחלקם בי"ז יהיו י"ז וי"א חלקים מי"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולדעת החלק האמצעי אמור אם בעת שהמחובר הוא י"ז שוה חלק האמצעי י"א בהיות המחובר ס' כמה הם חלקו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור ס"פ י"א הם תר"ס | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקם בי"ז יהיו ל"ח וי"ד חלקים מי"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולדעת חלקי הגדול אמור אם בעת שהמחובר הוא י"ז יהיה החלק הגדול י"ג בהיות המחובר ס' כמה יהיה חלקו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור ס"פ י"ג תש"פ | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקם בי"ז יהיו מ"ה וט"ו חלקים מי"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ויהיה סדר הענין כך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אומר הקטן אני שאני י"ז וי"א חלקים וחצי האמצעי שהם י"ט וז' חלקים מי"ז וחצי הגדול שהם כ"ב וי"ו חלקים מי"ז מחובר שלשתם ס' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואומר האמצעי אני שאני ל"ח וי"ד חלקים מי"ז ושליש הקטן שהם ה' וט"ו חלקים מי"ז ושליש הגדול שהם ט"ו וה' חלקים מי"ז מחובר שלשתנו ס' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואומר הגדול אני שאני מ"ה וט"ו [155]חלקים מי"ז ורביע הקטן שהוא ד' וז' חלקים מי"ז ורביע האמצעי שהוא ט' וי"ב חלקים מי"ז מחובר שלושתנו עולה ס' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שאלה יעקב חלק לבניו ג' והיה חלק ראובן גדול משל שמעון ושל שמעון גדול משל לוי נתן ראובן לשני אחיו מחלקו כפי מה שהיה חלקם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור כי חלק הראשון היה ד' וחלק השני ז' וחלק השלישי י"ג ונמצא בסוף חלקם שוה והיה ח' פשוטים לכל א' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כיצד דרך משל חלק ראובן היה י"ג וחלק שמעון ז' וחלק לוי ד' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה התחיל ראובן ונתן מחלקו לשמעון ז' כפי שהיה לו וללוי ד' ושנים נשאר בידו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואח"כ שמעון שעכשיו היה לו י"ד נתן ללוי ח' כפי מה שהיה בידו ולראובן ב' כפי מה שהיה עכשיו בידו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואח"כ לוי שעכשיו היה לו י"ו נתן מאלו לראובן ד' ונמצא שיש לו ח' וד' לשמעון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועם זה נמצא שביד כולם יש ח' פשוט' וחלקם שוה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Buy and Sell Problems | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אדם א' אמר הנה שני כלכלות של תאנים ובכל א' יש ק' תאנים משל היפות כ' לפשוט והרעות [156]ל' לפשוט עולה חשבונם ח' פשוטים ושליש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אדם שכר שכיר לל' יום בשכר [...] פשוט לכל יום והיה צריך לפרוע מידי יום ביום ולא היה לו כי אם ששה מטבעות ששוויים בין הכל ל' פשוטים ועכשיו שואלים מה יהיה שווי המטבעות כדי שיוכל לפרוע מידי יום ביומו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תשובה שיווי המטבעות צריך להיות כזה א' ב' ג' ד' ח' י"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Ordering Problem | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אדם היה לו זאב ועז וכרוב והיה לו לעבור מעבר מים ולא היה כי אם ספינה קטנה מאד שאינה מחזיק כי אם האדם ודבר אחד ולא יותר ואם תניח העז והכרוב ויעביר הזאב העז אכל הכרוב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תחלה יעביר העז ויניח הזאב והכרוב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואחר יעביר הכרוב ויחזור להעביר העז עמו [157]למקום הראשון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ויעביר הזאב לעבר האחר ויחזור להעביר העז עמו לעבר הא' ויעביר הכרוב ויניח העז ואחר יחזור ליקח העז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Joint Purchase Problem - "If You Give Me" | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ראובן אמר לשמעון אם אתה נותן לי אלף זהובים יעלה הקרן שלי כסכום מעותיך ושמעון משיב לראובן ואם אתה נותן לי אלף זהובים אני אכפול מעותי לסכום מעותיך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תשובה ראובן יש לו ה' אלפים זהובים ושמעון יש לו ז' אלפים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה פי' ראובן שואל לשמעון אלף זהובים ואם שמעון יתן לו נשאר ביד שמעון ו' אלפים וראובן שהיה לו ה' אלפים תחלה עכשיו עם האלף שנותן לו שמעון יש לו ו' אלפים נמצא שלכל א' יש להם ו' אלפים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ושמעון אומר לראובן אם אתה תתן לי אלף זהובים נמצא שישאר ביד ראובן ד' אלפים והקרן של שמעון שהיה ז' אלפים נמצא עכשיו עולה לסכום ח' אלפים והיינו הכפל ממה שנשאר ביד ראובן | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Chapter On Cubic Roots [P1088 2r-v] |
והילך שער על דבר מרובע על כל עבריו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כגון שתחשוב חוה פעם חוה וזהו רבוע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כמו שתאמר ב פעמים ב' זהו ד' כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תאמר ותחשוב הד' חתיכות גם ב' פעמים לעובין ויהיו ח' זהו רבוע על כל עבריו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ב' פעם ב' זהו ד' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ב' פעמים ד' זהו ח' כו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כשתחשוב דוד פעם דוד עולה וטבהאב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תאמר דוד פעם וטבהאב ועולה דדגזטחטט כו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דומיון הנה הכלל העולה מן החשבון המרובע לכל צדדיו הוא כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותמנה לעולם ברביעיות כגון אטבא אבזט טדא ומה שלא עולה לרבעיות מאותן אותיות תוכל להבין החשבון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עתה בכלל הנרשם טדא ג' אותיות ותאמר מה הריבוע המרובע על כל עבריו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועתה הסר הבא מן טדא ונשאר ד"ב ויהא כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותכתוב ה' תחת הד' ועל הד' כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומעתה תשלש האות הנמצא ר"ל ה' ונסוג שלש מעלות לאחריך דהיינו מן ה' יהיה ה"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותכתוב ה' תחת ב' וא' תחת ז' כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועתה תעיין מה אות מצורף לאות ה' שממנה בא זה החשבון הכלל הנרשם ואז תנסה ותצרף אליה אות שאם תחשוב כאשר אשכילך שיהא מכוון אות האחרון מן חשבון העולה לאות אחרון מן חשבון אשר אנו מתעסקים בו או בקרוב דומה לאות אחרון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועתה הילך הדרך תלך בו תקח ג' וכתוב למעלה על הא' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועתה נסה אם אמת הדבר תצרף ג"ה של מעלה עם ה"א של מטה ויעלה הטז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועתה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואז תוסיף | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה ה' אותיות תשים נגד ה' אותיות הכלל הנרשם דהוא כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותחסר כלל התחתון מן העליון ונשאר כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועתה יהיה החשבון צורתו כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועתה נסוג ג' עם הא' ג' מעלות לאחריך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונסוג ג' ג"כ לאחריך ג' מעלות ותשלש ויהיה ט' וצורתו כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועתה תאמר מה היה אות ראשון ע"כ היה א' כי לעולם כשיהיה א' אות ראשון מן הכלל העולה היה הפרט א' ואם אות ראשון ח' אז היה הפרט ב' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועתה תחשב אג"ה עם טה"א ויעלה טבדדח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועתה תצרף הא' ג"כ עם כל אות אטבדד"ח ותשים לפניו א' פעמים א' ויהיה כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נמצא שהם מכוונים זה העולה מן אג"ה פעמים טה"א אחד צרופך והכלל הנרשם למעלה ולעולם כשהם מכוונים אז החשבון אמת ואם יוותר לך אפי' אות אחת תוכל להבין שלא [נאות] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דומיון אחר הכלל העולה ד ד ג ז ט ח ט ט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נתחיל מאות ט' הראשונה כי היא אות רביעית ונקח מן ט"ט הריבוע והוא ע"כ ד' כי לא תוכל ליקח יותר והוא ד' פעמים ד' עלה ד"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חסר ד"ו מן ט"ט ונשאר ה"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אותו ד' תכתוב למטה תחת הט' ולמעלה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נסוג ד' ג' מעלות ותשלש לאחריך ויהיה ב"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תכתוב ב' תחת הט' וא' תחת ח' כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואח' תצרף אל הד' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תצרף אליו ה' אז אינו מכוון פרט העולה לחשבון הכלל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולפעמים לפי האמת אינו מכוון אך תדקדק על אות הסמוך לאות אחרון שהוא דומה קצת | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועתה קח ו' ותצרף לד' ותאמר ו"ד פעמי' ב"א ויעלה בה"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תצרף ו' עם בה"ה ויעלה כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ושים עליו ו' פעמים ו' ויהיה כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תחסר זה העולה מן ה' אותיות החשבון הנרשם ויהיה אחר חסרונך כזה ועתה צורתו כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועתה נסוג עם ו' העולה ג' מעלות לאחריך ותשלש וב"א ג"כ ג' מעלות לאחריך ויהיה כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועתה מה היה אות ראשון ע"כ ד' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותחבר דוד עם חג"א ויעלה כו' ושים עליו ד' פעמי' ד' ויעלה הפרט אות באות כגון הכלל ודוק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Here is a Chapter, Called the Chapter on Square Roots [P1088 6v-7v] |
הילך שער ונקר' שער השורש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| ושייך על דבר כשחשבת ריבוע | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כגו' חו"ה פעם חו"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
או זה"ב פעם זה"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שתראה מה תחילת החשבון אע"ג שלא ידעת בתחילה הפרט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועת' אכתו' דומיון חשבתי דבר ועולה ממנה כזה הוה הכלל
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וראה מה היה מתחילה הפרט שעולה ממנ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועתה אשכילך ותן לחכם ויחכם עוד ויסף לקח[note 11] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
קח הכלל לפניך ואראה לך דבר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
לעולם תתחיל מן האו' שלא מכוון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כגו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם האותיו' ח' אז תתחיל באות ז' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן לעולם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם האותיו' ז' אז תתחיל באות האחרון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועתה אראה חשב' הכלל ו' אותיות ו"טב"הא"ב אז תתחיל באו' ה' וזהו א' ותאמ' מה הריבוע בא"ב פי' מה אות אתה יכול ליקח זה פעם זה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אי אתה יכול ליקח ה' פעם ה' מן א"ב כי ה' פעם ה' זהו כ"ה והשו' אין לך אלא כ"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אלא אתה יכול [לומ'] ליקח ד' פעם ד' כי ד' פעם ד' זהו ו"א פי' י"ו ועוד נשאר לך ה' ואות' ד' העולה כי הריבוע בא"ב זהו ד' פעם ד' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואות' ד' תכתוב על הא' מן ו'ט'ב'ה'א'ב' ותחת הא' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותמחק הא"ב מן וטבהא"ב ותכתוב במקומ' ה' כי מן ד' פעם ד' נשאר ה' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והד' תכתו' למעלה מן הה' ולמט' מן הה' והילך צורתו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואז תכפול הד' הריבוע העומד [ותמשוך] הח' לאחריה תחת ה' השנייה ויהא ח' והד' העומדת על הה' תמשוך ג"כ לאחריה על ה' השניי' אבל לא תכפול אות' וצורתו כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואז תאמר כמה פעם ח' בה"ה פי' בנ"ה והוה בה ו' פעמים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אות' ו' תכתוב למעלה מן הב' ולמטה מן הב' כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועוד נשאר ז' וז' מן ה"ה ותמחוק השני ה' ותכתו' במקומ' הז' ויהא צורתו כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והו' העולה מן השני ה' העומדת על הב' ולמט' מן הב' תקח אות' נמי ו' פעמי' ו' פעם ו' זהו ו"ג פי' ל"ו וקח הו"ג מן הב"ז קח ארבעי' מן הע' כי הב"ז הוה ע"ב ועוד נשאר ב' ודע שלקחת ארבעי' ולא אתה צרך אל צריך אלא ל"ו אז קח הד' מן המ' ושים אות' על הב' שלפני הז' ויהא צורתו כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועתה תכפול הו' ג"כ לאחריה ויהא י"ב ואז תכתו' הכי שים הב"א לאחריה והח' נמי לאחרי' ואז תעשה הכי שים הב' תחת הט' והא' שים על הח' ויהיה ט' ותשים נמי למפריע הו' העליונ' ולא תכפול ושי' אות' על הט' והד' העליונ' תמשוך נמי לאחריה על הו' נגד הט' כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואז תאמ' כמה פעמים ט' בו"ג פי' בל"ו והוה בו ד' פעמים ולא נשארי' לך מאומה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואות' ד' תכתו' על הו' הראשונ' ותחת הו' ותמחק הו"ג של מעלה והט' של מטה וצורת' כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואז קח הב' נמי ד' פעמים מן הט' ועוד נשאר לך א' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונמח' הט' ותכתוב במקו' א' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונמח' הב' תחתונ' כי לקחנו נמי ד' פעמי' ועוד נשאר כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואז קח הד' נמי ד' פעמי' מן ו"א פי' מן י"ו ואז לא נשאר לך מאומה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נמצא שהפרט היה בתחילה דו"ד פעם דו"ד ועולה הכלל ו"טב"הא"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Always know that when you do it this way and you have nothing left, then the result you received is the original number. | ודע לעולם כשתעשה בדרך זה ולא נשאר לך מאומ' אז מה שעולה בידך זה היה תחילת החשבון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועוד אראה לך דומיון החשבון עולה כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אז תתחיל בט' כי הט' אות חמיש' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואז תאמר כמה הריבוע בט' ודע שהריבוע בט' הוה ג' כי ג' פעמי' ג' זהו ט' ולא נשאר לך מאומה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והג' תכתוב תחת הט' ועל הט' כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואז תכפול הג' לאחריה והוה ו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותשים לאחריה תחת הז' והג' שלמעלה תמשוך לאחריה על הז' אבל [לא] תכפול כמו שתעשה לג' תחתונ' והילך צורתו ונמחק הט' כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואז תאמ' כמה פעמי' ו' בז' ואינ' אלא פעם א' ועוד נשאר א' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואות' א' שעולה מן הז' תכתוב על הג' שלפני הז' ותחת הג' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונמח' הז' ותכתו' במקומ' א' הנשארת מן הז' שלא מגיע נמי לז' וזה וצורת' כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואז תקח הא' נמי א' פעם מן ג"א ועדיין הוה ב"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואז תכפול הא' שתחת הב' לאחריה תחת הד' ויהא ב' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והו' תשים ג"כ לאחרי' אבל לא תכפול שכבר כפלת הו' ותשים אות' ו' תחת הב' שכבר עומד שם הא' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והא' למעלה על הב' נמי תמשוך לאחריה על הד' ולא תכפול והג' במקו' הא' על הב' כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואז תאמר כמה פעמים ו' בב"א פי' בי"ב והוה בו ב' פעמים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אות' ב' תכתוב על הד' הראשונ' ותחת הד' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותמחוק הב"א ויהא צורתו כזה והו' שלמט' נמי תמחוק ויהא כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואז תקח הב' נמי ב' פעמים והוה ד' ולא נשאר מאומ' והב' השנייה נמי תקח ב' פעמים מן הד' ואז לא נשאר לך מאומא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נמצא הפרט הוה ב'א'ג' פעם ב'א'ג' ויוצא ממנ' כלל ד'ד'ג'ז'ט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| ודוק ותובין לעולם בדרך זה | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Notes
Apparatus
| |
Appendix I: Glossary of Terms
| to know | לידע, דע כי, דע שתדע ש |
| to explain | לפרש, ביארתי |
| chapter | שערים |
| teaching | הקבלות |
| one who calculates | המחשב, המפתח |
| calculation | החשבונות |
| numeral | חשבון, אותיות, ציפרא |
| zero | גלגל |
| place holder | שומר המעלות |
| first rank | מעלה הראשונה |
| units | מעלת היחידים |
| third rank | מעלה השלישית |
| hundreds | מעלת המאות |
| fourth rank | מעלה הרביעית |
| thousands | מעלת האלפים |
| fifth rank | מעלה החמישית |
| tens of thousands | מעלת הרבבות, י' אלפים |
| sixth rank | מעלה ששית |
| hundreds of thousands | מעלת מאה אלפים |
| seventh rank | מעלה שביעית |
| millions | מעלת אלף אלפים |
| smaller than | קטן מן |
| greater than | גדול מן |
| more than | יותר מ |
| to exceed by | יותר על, יתיר על, עודף על, למעלה מ |
| bottom numeral | התחתונה, תחתונה |
| upper numeral | העליונה, עליונה |
| infinitely, endlessly | עד אין מספר |
| times | פעמים |
| to multiply | לחשוב, לכפול זע"ז |
| rank | מעלות, מעלה |
| המעלה שנייה | |
| עליונה | |
| שיט' | |
| שבשיט' עליונ', שיטות עליונות, שיטות העליונות | |
| שיט' ראשונ' | |
| השיטה התחתונה | |
| דומיון, דמיון | |
| מגיע ל | |
| עולה, יעלה, יעלה בידך, מה שיעלה בידך, העולה בידך | |
| יצא | |
| נקראת, נקרא | |
| יקראו | |
| אות, האות | |
| מניינם, מניין | |
| תחשוב | |
| חשוב | |
| חשוב אותו | |
| חשבונך | |
| תאמר, אמור | |
| ישאלך | |
| שואל | |
| תשיב אל לבך | |
| לכתוב, כתוב, כתוב (אותו / אותה / ב), כתבתי, כתבת, תכתוב (אותו), נכתוב, אכתוב לך, כותבין | |
| כתיבה | |
| שכתבת | |
| רשום, ארשום לך | |
| חרו[ת]ים | |
| העתקתי | |
| לראות | |
| שאראך | |
| אדירין | |
| חברם יחד | |
| לחבר, לחבר ... עם, תחבר...אל, תחבר... על, תחבר... ה, חבור... על, חבור אל ה, נחבר... עם, נחבר... על, אחבר | |
| לחבר כמה חשבונות יחד | |
| חבורם, חבורו, חיבור שחברנו | |
| הטור מן החיבור | |
| צירופו | |
| תצרף, צרוף (...יחד), צורפם יחד, צרפם (יחד) | |
| נוסיף... על, נוסיף... עליה | |
| מוסיף ...על, מוסיף... עליה | |
| בשער החיבור, שער חיבור | |
| המחובר | |
| השליך, השלך (...מ / ה), השלך מידך, קח ממנו, תקח ... מן, קח מה ש | |
| השלך ט"ט... מ, השלך ... בט"ט | |
| נשאר, ישאר, ישאר בידך, ישאר לך, הנשארת | |
| בלי חסרון ויתרון | |
| ומה שנשאר לך, ומה שנשמר לך | |
| הנותרת לי | |
| משקלו, משקל ה, משקלים | |
| אבני המשקל, אבן הראשון | |
| תכפול יחד, לכפול יחד | |
| תכפול | |
| כפל | |
| כפל קטן | |
| מה שבא מן הכפל | |
| בשער כפל | |
| לוח | |
| חצי לוח | |
| מתוקנת | |
| שער הכפל קטן, שער כפל קטן | |
| ציור | |
| צורתו | |
| עברת על ה, תעבור | |
| תקח ה, קח ה, קח בידך, קח בידך ל, יקח ה... מה | |
| ומהם תבין | |
| מרחק מן, המרחק עד, מרחקים | |
| מכל אחד, מן כל אחד ואחד | |
| זה על זה | |
| דהיינו, היינו | |
| הילך | |
| היאך | |
| המניין הקטון, מניין קטון, המנין הקטן, האות הקטן | |
| אות הגדול | |
| tens | העשיריות |
| ספר | |
| גלחות, בגלחת | |
| מחוק | |
| עניין | |
| כלל | |
| פי' | |
| המותר מ, עודף עליו | |
| לעשות, עשה, תעשה, עשיתי | |
| כאשר, כש | |
| עתה | |
| תדיר | |
| לעולם | |
| ולפעמים | |
| לא ... כלל | |
| לא ימוש מפיך | |
| תוכל ל | |
| כל כמה שתוכל | |
| אינך צריך | |
| צריך ל | |
| צריך ש | |
| תלוה לו, הלוית לו | |
| תרצה (ל), תחפוץ ל, בקשת ל, רצונך, בקשנו ל | |
| אשר בקשת, מבוקש | |
| מתכונך | |
| מכוון | |
| כוינת חשבונך | |
| אשכילך | |
| תשכיל, תחכם | |
| מושכל ראשון | |
| עיין | |
| תקיש | |
| ודוק | |
| להודיעך ש | |
| למצוא, תמצא (ה), נמצא, מצאתי, נמצא ה | |
| לזכרון | |
| וככלות | |
| תשלם | |
| המלאכה | |
| סליק | |
| הוי אומר | |
| בדרך הזה, דרך אחרת ל | |
| ואז, אז | |
| כבר | |
| כדי ש | |
| מה ש | |
| כל מה ש, כל אשר | |
| אשר ב | |
| אף כי | |
| כי | |
| לכך | |
| והנה | |
| נמי | |
| אחר כך, אח"כ | |
| ואם... אז | |
| אם | |
| אפילו | |
| רק | |
| כמה | |
| יהיה בכל פעם | |
| פעמים | |
| לכל היותר | |
| יותר מן | |
| מותר ה... מ | |
| פחות מ, פחותים מ, מה שלמטה מן, למטה מ | |
| הרבה כל כך | |
| ויהיה, ויהי, ויהא | |
| שם | |
| יש | |
| הרי | |
| ויהיה הכל | |
| הוה | |
| שהם | |
| אני | |
| הוא, היא | |
| או כלך לדרך זו, או כלך בדרך זו | |
| זה, זה ה | |
| כזה | |
| הזה | |
| אילו ה | |
| האחרונים | |
| חשבונות, חשבון | |
| שבידך | |
| בא לידך מ | |
| לפניך, מה שלפניך | |
| הסמוכ' לה, הסמוך ל | |
| מצד אותיות של שמאל | |
| לצד שמאל, בצד שמאל (ל), לשמאל | |
| אצל, אל | |
| נגד | |
| במקום | |
| אחר | |
| עוד | |
| ועוד | |
| וכן, ג”כ | |
| וכפי זה | |
| כגון | |
| כאילו | |
| כמו כן | |
| כל כיוצא בזה | |
| שלאחריו, לאחריה, שלאחריה | |
| שלפניה, לפני ה, קודם ה | |
| מן ה | |
| תחת, תחת ה | |
| למטה, שלמטה | |
| למעלה, למעלה מזו | |
| לעיל | |
| שאין בו ממש | |
| בקלות | |
| ולא ... אלא | |
| בלא שום | |
| זכר ל | |
| דבר | |
| כל דבר | |
| את"ל | |
| בע"ה | |
| לישב על אופניו |
Appendix II: Bibliography
Anonymous
Sefer Ṣifra
Manuscripts:
- 1) Budapest, Magyar Tudományos Akadámia, Ms. Kaufmann A 520/10 (IMHM: f 15170), ff. 179-200; 217-218 (16th century)
- Kaufmann A 520/10
- 2) Cincinnati, Hebrew Union College 890/1 (IMHM: f 30901), ff. 4r-18v (Hanau, 1641)
- Cincinnati 890
- 3) Mantova, Comunità Ebraica MS ebr. 8/16 (IMHM: f 788), ff. 69r-71v (Porto, 1464-1471)
- Mantova 8
- 4) Moscow, Russian State Library, Ms. Guenzburg 714/4 (IMHM: f 27994), ff. 64r-83v (17th-18th century)
- Guenzburg 714
- 5) München, Bayerische Staatsbibliothek, Cod. hebr. 394/4 (IMHM: f 1223), ff. 109v-127r (1566)
- München Cod. hebr. 394
- 6) New York, Jewish Theological Seminary Ms. 2634/6 (IMHM: f 28887), ff. 94r-124v
- JTS 2634
- 7) Oxford, Bodleian Library MS Mich. 60/11 (IMHM: f 22085), ff. 151r-176v (cat. Neub. 2171); (Frankfurt am Main, 1537)
- 8) Oxford, Bodleian Library MS Mich. 440/4 (IMHM: f 19984), ff. 114v-124v (cat. Neub. 2170); (1645)
- 9) Paris, Bibliothèque Nationale heb. 1088/1 (IMHM: f 14921), ff. 1r-23v (15th century)
- heb.1088
- 10) Paris, Séminaire Israélite de France (École Rabbinique) 158/1 (IMHM: f 4102), ff. 197r-211v (1604)
- École Rabbinique 158
- 11) Warszaw, Żydowski Instytut Historyczny 288/1 (IMHM: f 12013), ff. 1r-5v (15th century)
- 12) Warszaw, Żydowski Instytut Historyczny 295/4 (IMHM: f 10129), f. 56v (16th century)
The transcript is based mainly on manuscript München 394
Bibliography:
- Steinschneider, Moritz (Moshe). 1893–1901. Die Mathematik bei den Juden. Berlin-Leipzig-Frankfurt: Kaufmann; repr. Hildesheim: G. Olms, 1964 and 2001. pp. 216-217 [i478-i479]
- ———. 1905. Mathematik bei den Juden, Band II: 1551-1840. Monatsschrift für die Geschichte und Wissenschaft des Judenthums 49, pp. 85-86. repr.: ed. Gad Freudenthal, Hildesheim, Zürich, New York: Olms, 2014, pp. 8-9.
![\scriptstyle{\color{OliveGreen}{x,y<10\quad x\sdot y=\left[10\sdot\left[\left(x+y\right)-10\right]\right]+\left[\left[x-\left[\left(x+y\right)-10\right]\right]\sdot\left[y-\left[\left(x+y\right)-10\right]\right]\right]}}](/mediawiki/images/math/8/4/f/84fd76022cd8069c30df21ae18e6c87c.png)
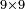
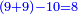


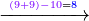
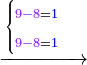
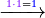
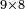
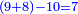


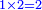
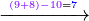
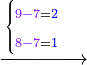
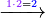
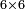
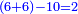

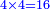

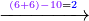
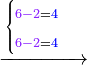
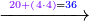
![\scriptstyle{\color{OliveGreen}{x,y<10\quad x\sdot y=\left[10\sdot\left[\left(x+y\right)-10\right]\right]+\left[\left(10-y\right)\sdot\left(10-x\right)\right]}}](/mediawiki/images/math/3/4/e/34ed2780428a539eca5ec2e8c8793406.png)



![\scriptstyle{\color{OliveGreen}{x<y<10\quad x\sdot y=\left(x\sdot10\right)-\left[x\sdot\left(10-y\right)\right]}}](/mediawiki/images/math/6/3/7/6374cb483753892c8270803768cbdd35.png)

![\scriptstyle{\color{blue}{7\times8=\left(7\sdot10\right)-\left[7\sdot\left(10-8\right)\right]=70-\left(7\sdot2\right)=70-14=56}}](/mediawiki/images/math/c/f/8/cf82d668175564f785f54108257ef5e3.png)



![\scriptstyle{\color{OliveGreen}{x+y<10\quad x\sdot y=10+\left[\left(x-1\right)\sdot\left(y-1\right)\right]-\left[11\left(x+y\right)\right]}}](/mediawiki/images/math/d/5/d/d5d3c8b350120abb208207c03e53e9e3.png)

![\scriptstyle{\color{blue}{4+4+\left[11-\left(4+4\right)\right]=4+4+3=11}}](/mediawiki/images/math/b/4/2/b42c664ab942373f7f7104da31b46a3d.png)
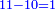
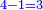


![\scriptstyle\xrightarrow{{\color{Purple}{\left[4+4+\left[11-\left(4+4\right)\right]\right]-10}}={\color{blue}{1}}}](/mediawiki/images/math/6/1/e/61e489ce126e7bf73a517c1a9df4564b.png)
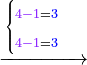
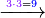
![\scriptstyle\xrightarrow{{\color{Purple}{9-\left[11-\left(4+4\right)\right]}}={\color{blue}{6}}}](/mediawiki/images/math/9/6/f/96fe370a4741feb5cff3d537a3aa701a.png)
![\scriptstyle{\color{OliveGreen}{x+y<10\quad x\sdot y=\left[\left(10-y\right)\sdot\left(10-x\right)\right]-\left[10\sdot\left[10-\left(x+y\right)\right]\right]}}](/mediawiki/images/math/c/0/7/c079212d57efd40f34bffb4b6570833f.png)
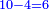

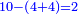
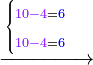

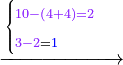
![\scriptstyle{\color{OliveGreen}{x+y<10\quad x\sdot y=\left[\left(10-y\right)\sdot\left(10-x\right)\right]+\left[10\sdot\left(x+y\right)\right]-\left(10\sdot10\right)}}](/mediawiki/images/math/3/5/0/350e44e97c9ca33b70b02f10b6b59ff2.png)
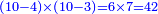
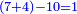

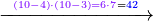

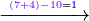
![\scriptstyle{\color{OliveGreen}{x,y<10\quad x\sdot y=\left[\left(10-y\right)\sdot\left(10-x\right)\right]+\left[10\sdot\left[\left(x+y\right)-10\right]\right]}}](/mediawiki/images/math/8/4/c/84c94515c6f1ba076a08feee4671e7ff.png)
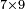

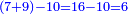
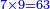
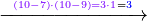

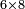
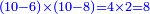
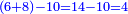

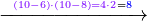
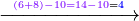
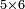

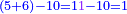

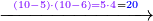
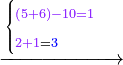



 :
: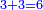
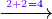
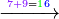
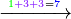



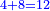
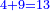

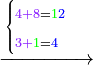
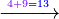



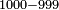
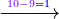
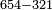
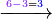
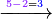


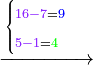
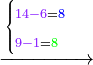
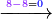
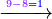
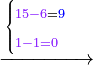
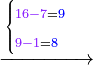
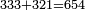



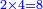

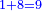

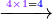
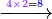
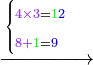
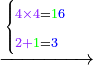

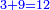
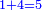

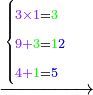


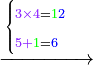

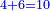

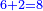

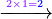



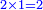

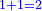
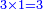
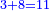
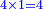
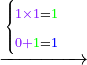

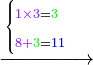




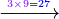
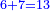

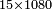
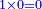

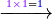
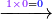
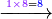



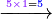
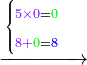
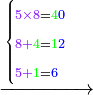


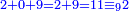
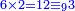

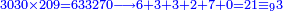
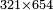
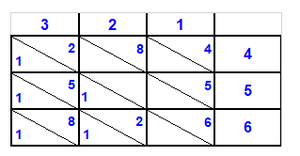

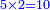

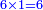
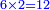

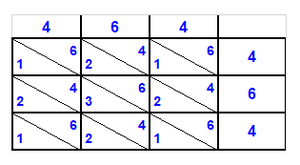
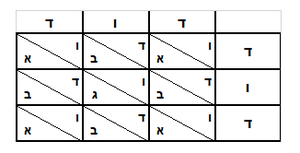
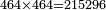
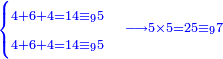
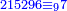


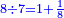
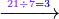
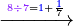
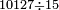
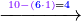


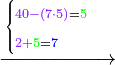
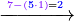
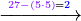
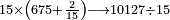
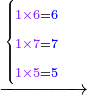


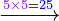
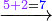
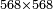

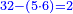

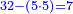
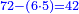

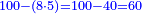
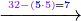
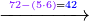

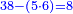
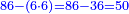


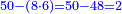
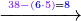
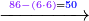
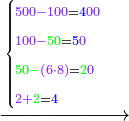
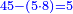
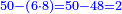

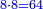
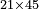
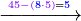

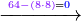
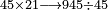

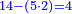

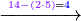
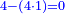

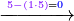
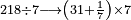

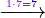
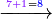


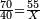


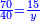
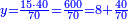
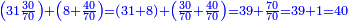
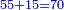
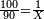

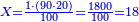
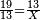




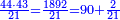
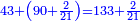
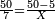
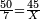

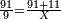

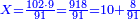
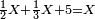
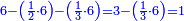
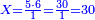
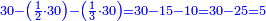
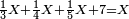




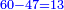

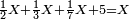
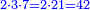
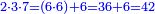
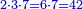

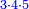
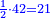

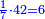
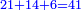
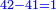
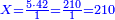

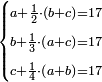
 ].
]. ].
].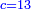 ]
]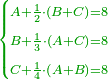
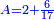

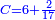
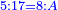

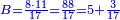



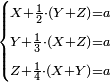

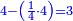
![\scriptstyle{\color{blue}{\left(4+\frac{1}{2}\right)-\left[\frac{1}{3}\sdot\left(4+\frac{1}{2}\right)\right]=3}}](/mediawiki/images/math/b/2/c/b2c7654e9e36456843cf38a9cefba0f9.png)
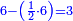
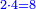
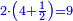
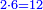

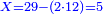
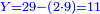
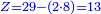
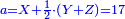
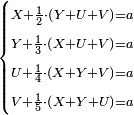
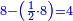
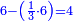
![\scriptstyle{\color{blue}{\left(5+\frac{1}{3}\right)-\left[\frac{1}{4}\sdot\left(5+\frac{1}{3}\right)\right]=4}}](/mediawiki/images/math/2/f/8/2f8b0ce274ed2357653f5bbb5b2e2059.png)
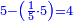
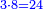
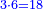

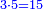
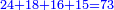

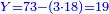
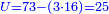
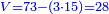
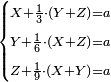
![\scriptstyle{\color{blue}{\left(3+\frac{3}{5}\right)-\left[\frac{1}{6}\sdot\left(3+\frac{3}{5}\right)\right]=3}}](/mediawiki/images/math/d/b/8/db875db8eb50eca9108eb5f305313319.png)
![\scriptstyle{\color{blue}{\left(3+\frac{3}{8}\right)-\left[\frac{1}{9}\sdot\left(3+\frac{3}{8}\right)\right]=3}}](/mediawiki/images/math/7/f/1/7f1cf2e5edc0dd92725597fe77ab6fbe.png)
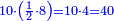


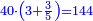
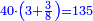

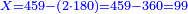
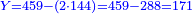
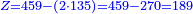

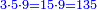
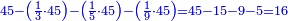
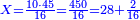
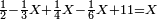
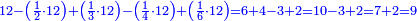
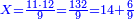
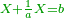
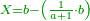
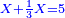
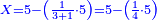
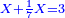
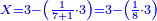
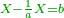



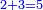



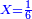
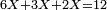
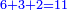
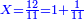
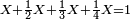
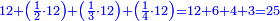
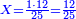
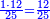
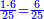
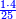
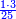
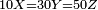
![\scriptstyle{\color{OliveGreen}{\begin{cases}\scriptstyle1+\left[3\sdot\left[10-\left(1\sdot7\right)\right]\right]=1+\left[3\sdot\left(10-7\right)\right]=1+\left(3\sdot3\right)\\\scriptstyle4+\left[3\sdot\left[30-\left(4\sdot7\right)\right]\right]=4+\left[3\sdot\left(30-28\right)\right]=4+\left(3\sdot2\right)\\\scriptstyle7+\left[3\sdot\left[50-\left(7\sdot7\right)\right]\right]=7+\left[3\sdot\left(50-49\right)\right]=7+\left(3\sdot1\right)\end{cases}}}](/mediawiki/images/math/9/e/a/9eae4ff1a7d19532b4c04b80bbf4a91a.png)


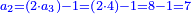

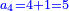
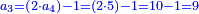
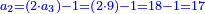
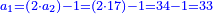
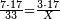

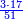
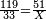
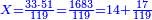
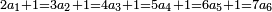
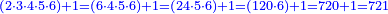
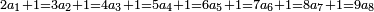
![\scriptstyle{\color{blue}{\left[720\sdot\left(7\sdot8\right)\right]+1=\left(720\sdot56\right)+1}}](/mediawiki/images/math/4/7/7/4771f98330aab4d54b098ef66e8365c0.png)
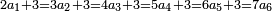
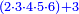


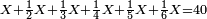
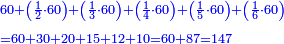

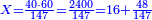
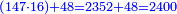
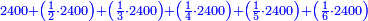
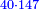
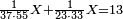
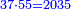

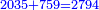


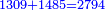

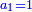


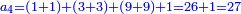
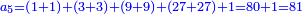
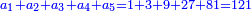
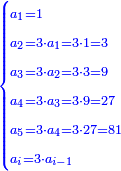
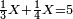
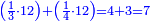
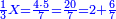
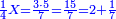

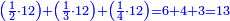
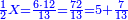
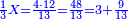
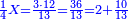
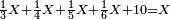
![\scriptstyle{\color{blue}{60-\left[\left(\frac{1}{3}\sdot60\right)+\left(\frac{1}{4}\sdot60\right)+\left(\frac{1}{5}\sdot60\right)+\left(\frac{1}{6}\sdot60\right)\right]=60-57=3}}](/mediawiki/images/math/a/f/0/af01839e49ad14fc9850f6d812f5b8cb.png)
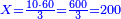
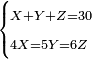
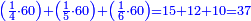
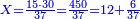
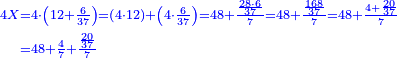
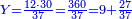

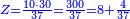
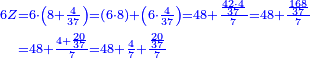
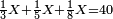

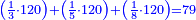
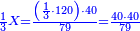
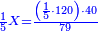
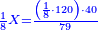
![\scriptstyle\begin{cases}\scriptstyle\sum_{i=1}^4 a_i =40\\\scriptstyle a_1=\left(\frac{1}{2}+\frac{1}{6}\right)\sdot a_2\\\scriptstyle a_2-\left[\left(\frac{1}{2}+\frac{1}{6}\right)\sdot a_2\right]=a_2-a_1=\frac{2}{3}a_3\\\scriptstyle a_3=\left(\frac{1}{3}+\frac{1}{4}+\frac{1}{6}\right)\sdot a_1\\\scriptstyle a_4=\frac{4}{5}\sdot{\color{red}{a_3}}\end{cases}](/mediawiki/images/math/c/1/e/c1e254f07692c6845aa08397f170e7e7.png)
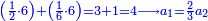

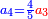
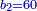
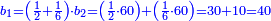
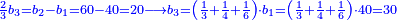
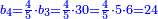
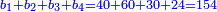
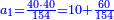
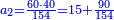

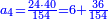
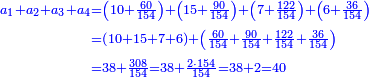
![\scriptstyle1+\left[\frac{1}{10}\sdot\left(X-1\right)\right]=2+\frac{1}{10}\sdot\left[X-\left[\left[1+\left[\frac{1}{10}\sdot\left(X-1\right)\right]\right]+2\right]\right]](/mediawiki/images/math/0/4/e/04ec9fa2f7109a05d7c4e8fa7ce5d879.png)
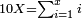
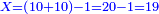

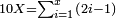
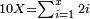
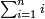
![\scriptstyle{\color{blue}{\sum_{i=1}^9 i={\color{OliveGreen}{\left[\frac{1}{2}\sdot\left(9+1\right)\right]\sdot9}}=5\sdot9=45}}](/mediawiki/images/math/6/f/0/6f09e82bc29722bd17b0356389427645.png)
![\scriptstyle{\color{blue}{\sum_{i=1}^{10} i=\left[\left(\frac{1}{2}\sdot10\right)\sdot10\right]+\left(\frac{1}{2}\sdot10\right)=\left(5\sdot10\right)+5=50+5=55}}](/mediawiki/images/math/8/0/b/80b5dca53c99289503b8c13b342ab214.png)
![\scriptstyle n=2m\longrightarrow\sum_{k=1}^n k=\left[\left(\frac{1}{2}\sdot n\right)\sdot n\right]+\frac{1}{2}n](/mediawiki/images/math/a/8/a/a8a5babcd3a6eea49bd9b762c7395e9b.png)
![\scriptstyle n=2m+1\longrightarrow\sum_{k=1}^n k=\left[\frac{1}{2}\sdot\left(n+1\right)\right]\sdot n](/mediawiki/images/math/1/e/4/1e4ea38423bb671340fbd2275e275e63.png)
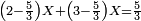

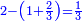
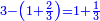
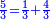
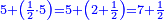
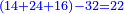

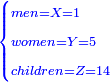

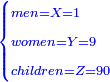
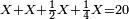
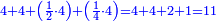

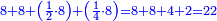

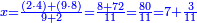
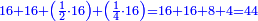

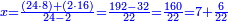
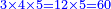
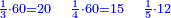
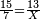
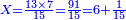
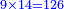

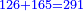

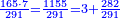
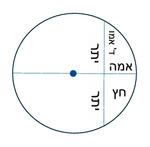
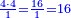


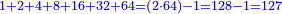

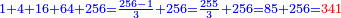
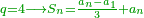
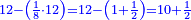



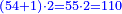
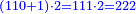
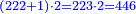



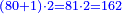
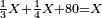



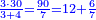
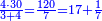

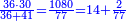
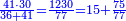
![\scriptstyle\left[\left(2-\frac{5}{3}\right)\sdot X\right]+\left[\left(3-\frac{5}{3}\right)\sdot X\right]=5](/mediawiki/images/math/b/3/9/b39a89f5f9f01ae578ff87165913ffb8.png)
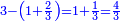
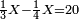


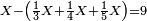
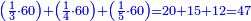
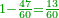
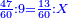
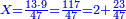
![\scriptstyle\begin{cases}\scriptstyle X+\left[\frac{1}{2}\sdot\left(Y+Z\right)\right]=60\\\scriptstyle Y+\left[\frac{1}{4}\sdot\left(X+Z\right)\right]=60\\\scriptstyle Z+\left[\frac{1}{3}\sdot\left(X+Y\right)\right]=60\end{cases}](/mediawiki/images/math/f/7/e/f7ed4efec08d6ecaa27c9fc465bdae6d.png)
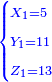
![\scriptstyle{\color{blue}{\begin{cases}\scriptstyle X_1+\left[\frac{1}{2}\sdot\left(Y_1+Z_1\right)\right]=17\\\scriptstyle Y_1+\left[\frac{1}{4}\sdot\left(X_1+Z_1\right)\right]=17\\\scriptstyle Z_1+\left[\frac{1}{3}\sdot\left(X_1+Y_1\right)\right]=17\end{cases}}}](/mediawiki/images/math/9/7/d/97d57b8ac4e8a9dbe8f698d6b961bdee.png)
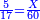
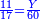
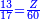
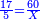

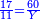
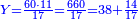
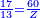
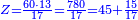
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle X+\left[\frac{1}{2}\sdot\left(Y+Z\right)\right]&\scriptstyle=\left(17+\frac{11}{17}\right)+\left[\frac{1}{2}\sdot\left[\left(38+\frac{14}{17}\right)+\left(45+\frac{15}{17}\right)\right]\right]\\&\scriptstyle=\left(17+\frac{11}{17}\right)+\left(19+\frac{7}{17}\right)+\left(22+\frac{16}{17}\right)=60\\\end{align}}}](/mediawiki/images/math/1/1/5/1151c1953e5eeefb1128f4a9ae687afb.png)
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle Y+\left[\frac{1}{3}\sdot\left(X+Z\right)\right]&\scriptstyle=\left(38+\frac{14}{17}\right)+\left[\frac{1}{3}\sdot\left[\left(17+\frac{11}{17}\right)+\left(45+\frac{15}{17}\right)\right]\right]\\&\scriptstyle=\left(38+\frac{14}{17}\right)+\left(5+\frac{15}{17}\right)+\left(15+\frac{5}{17}\right)=60\\\end{align}}}](/mediawiki/images/math/8/c/5/8c51fcde67c49f9f297096f4fcd9e029.png)
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle Z+\left[\frac{1}{4}\sdot\left(X+Y\right)\right]&\scriptstyle=\left(45+\frac{15}{17}\right)+\left[\frac{1}{4}\sdot\left[\left(17+\frac{11}{17}\right)+\left(38+\frac{14}{17}\right)\right]\right]\\&\scriptstyle=\left(45+\frac{15}{17}\right)+\left(4+\frac{7}{17}\right)+\left(9+\frac{12}{17}\right)=60\\\end{align}}}](/mediawiki/images/math/5/a/2/5a2cd999d16458b3c413355c1586917a.png)
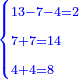


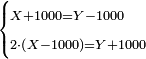
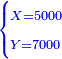





![\scriptstyle{\color{blue}{\sqrt[3]{149721291}}}](/mediawiki/images/math/2/8/8/28889c07ec72df83ee96c8d036f89e7d.png)

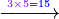
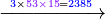
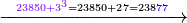
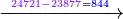
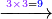
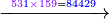
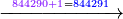
![\scriptstyle{\color{blue}{\sqrt[3]{99897344}}}](/mediawiki/images/math/7/3/e/73e3d56a32f3f45e418253824810fdba.png)
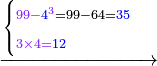
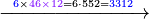
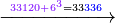


![\scriptstyle\xrightarrow{{\color{Purple}{\left[\left[4\times\left(464\times138\right)\right]\sdot10\right]+4^3}}={\color{blue}{2561344}}}](/mediawiki/images/math/3/9/b/39bf9e96de0aed4211fce111e0b692c4.png)