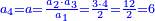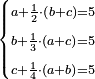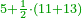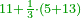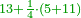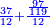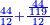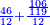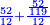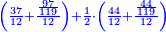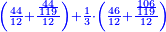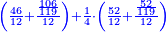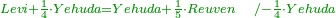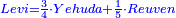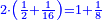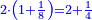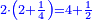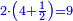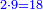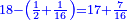Difference between revisions of "Excerpts Attributed to Immanuel Bonfils"
(→Extracting roots) |
(→Notes) |
||
| (152 intermediate revisions by the same user not shown) | |||
| Line 16: | Line 16: | ||
|style="text-align:right;"|<big>הקדמה</big> | |style="text-align:right;"|<big>הקדמה</big> | ||
|- | |- | ||
| − | |Know that the one is divided into ten parts called primes; each prime is divided into ten parts | + | |Know that the one is divided into ten parts called primes; each prime is divided into ten parts called seconds and so on endlessly. |
|style="text-align:right;"|<big>דע</big> כי האחד נחלק לעשרה חלקים יקראו ראשונים וכן כל ראשון נחלק לעשרה חלקים יקראו שניים וכן לאין תכלית | |style="text-align:right;"|<big>דע</big> כי האחד נחלק לעשרה חלקים יקראו ראשונים וכן כל ראשון נחלק לעשרה חלקים יקראו שניים וכן לאין תכלית | ||
|- | |- | ||
| Line 98: | Line 98: | ||
|style="text-align:right;"|ואם התחתון יותר גדול גרע שם העליון משם התחתון וכמספר שם הנשאר יפול החלוקה בהפך הצד ר"ל בשברים אם היו שניהם שלמים או בשלמים אם היו שניהם שברים | |style="text-align:right;"|ואם התחתון יותר גדול גרע שם העליון משם התחתון וכמספר שם הנשאר יפול החלוקה בהפך הצד ר"ל בשברים אם היו שניהם שלמים או בשלמים אם היו שניהם שברים | ||
|- | |- | ||
| − | |If one is integer and the other is fraction and the names of their ranks are the same, or not the same, add the names of their ranks and the quotient is as the name of the sum: fraction, if the upper is a fraction, or integer, if the upper | + | |If one is integer and the other is fraction and the names of their ranks are the same, or not the same, add the names of their ranks and the quotient is as the name of the sum: fraction, if the upper is a fraction, or integer, if the upper is an integer. |
:<math>\scriptstyle{\color{OliveGreen}{\frac{1}{10^n}\div10^m=\frac{1}{10^{n+m}}}}</math> | :<math>\scriptstyle{\color{OliveGreen}{\frac{1}{10^n}\div10^m=\frac{1}{10^{n+m}}}}</math> | ||
:<math>\scriptstyle{\color{OliveGreen}{10^n\div\frac{1}{10^m}=10^{n+m}}}</math> | :<math>\scriptstyle{\color{OliveGreen}{10^n\div\frac{1}{10^m}=10^{n+m}}}</math> | ||
|style="text-align:right;"|ואם האחד שלמים והשני שברים ושם מדרגותיהם שוה או בלתי שוה חבר שם המדרגות וכמספר שם העולה בשברים אם העליון שברים או בשלמים אם העליון שלמים שם יפול החלוקה | |style="text-align:right;"|ואם האחד שלמים והשני שברים ושם מדרגותיהם שוה או בלתי שוה חבר שם המדרגות וכמספר שם העולה בשברים אם העליון שברים או בשלמים אם העליון שלמים שם יפול החלוקה | ||
|- | |- | ||
| − | |Another Version - MS Paris 903 | + | | |
| + | === Another Version - MS Paris 903 === | ||
| + | |||
| | | | ||
|- | |- | ||
| − | | | + | |Know that the one is divided into ten parts called primes; each prime [is divided] into ten parts called seconds, each second into ten thirds, each third into ten fourths and so on endlessly. |
|style="text-align:right;"|דע כי האחד נחלק לי' חלקים יקראו שלמי'<sup>שברי'</sup> ראשוני' וכל אחד מהראשוני' לי' חלקי' יקראו שלמי' <sup>שברי'</sup> שניים וכל שניי' לי' שלישיי' וכל שלישיי' לי' רביעיי' וכן עד אין קץ | |style="text-align:right;"|דע כי האחד נחלק לי' חלקים יקראו שלמי'<sup>שברי'</sup> ראשוני' וכל אחד מהראשוני' לי' חלקי' יקראו שלמי' <sup>שברי'</sup> שניים וכל שניי' לי' שלישיי' וכל שלישיי' לי' רביעיי' וכן עד אין קץ | ||
|- | |- | ||
| − | | | + | |I call the rank of tens of integers "primes"; the hundreds of integers "seconds", the thousands of integers "thirds", the tens of thousands of integers "fourths", the [hundreds of thousands] "fifths" and so on endlessly. |
|style="text-align:right;"|וכן אני קורא מעלת העשרו' שלמים ראשונים ולמאות שלמי' שניי' ולאלפי' שלמי' שלשיי' ולרבואות שלמים רביעיי' ולרבבות חמשיי' וכן עד אין קץ | |style="text-align:right;"|וכן אני קורא מעלת העשרו' שלמים ראשונים ולמאות שלמי' שניי' ולאלפי' שלמי' שלשיי' ולרבואות שלמים רביעיי' ולרבבות חמשיי' וכן עד אין קץ | ||
|- | |- | ||
| − | | | + | |I call the rank of units by their name "units", since they are mean between the integers and the fractions, therefore, when the units are multiplied by units the result is units, which does not happen in any other rank. |
|style="text-align:right;"|אך האחדי' אני קור' בשמם אחדי' כי הם כאמצעיים בין השלמי' והשברי' על כן בכפל אחדי' באחדי' יצאו אחדי' מש"כ בשום מעל' אחרת | |style="text-align:right;"|אך האחדי' אני קור' בשמם אחדי' כי הם כאמצעיים בין השלמי' והשברי' על כן בכפל אחדי' באחדי' יצאו אחדי' מש"כ בשום מעל' אחרת | ||
|- | |- | ||
| − | | | + | |I call the seconds "having a greater name than" the primes, the thirds ["having a greater name than"] the seconds, and so on. This is for integers as well as for fractions. |
| − | |style="text-align:right;"|וכן אני קורא לשניי' גדולי השם מראשוני' וכן שלישיים משניי' וכן כלם בי' בשלמי' בי' בשברי' וכן באמ' חבר שם שניי' עם שם שלישיי' יצא חמשיי' וכן כלם | + | |style="text-align:right;"|וכן אני קורא לשניי' גדולי השם מראשוני' וכן שלישיים משניי' וכן כלם בי' בשלמי' בי' בשברי‫' |
| + | |- | ||
| + | |When I say: add the name of the seconds to the name of the thirds, the result is fifths and so on. | ||
| + | :<math>\scriptstyle{\color{blue}{10^{2+3}=10^5}}</math> | ||
| + | |style="text-align:right;"|וכן באמ' חבר שם שניי' עם שם שלישיי' יצא חמשיי' וכן כלם | ||
|- | |- | ||
| − | | | + | |Also, when I say: subtract the name of the primes from the name of the seconds; primes remain. |
| + | :<math>\scriptstyle{\color{blue}{10^{2-1}=10^1}}</math> | ||
|style="text-align:right;"|וכן באמ' גרע שם ראשוני' משם שניים ישאר ראשוני‫' | |style="text-align:right;"|וכן באמ' גרע שם ראשוני' משם שניים ישאר ראשוני‫' | ||
|- | |- | ||
| − | | | + | |If from the name of the primes, nothing remains, so they are in the rank of the units, for integers as well as for fractions. |
| + | :<math>\scriptstyle{\color{blue}{10^{1-1}=10^0}}</math> | ||
|style="text-align:right;"|ואם משם ראשונים לא ישאר דבר ויכפול במעל' האחדי' בי' בשלמי' בי' בשברי‫' | |style="text-align:right;"|ואם משם ראשונים לא ישאר דבר ויכפול במעל' האחדי' בי' בשלמי' בי' בשברי‫' | ||
|- | |- | ||
| − | | | + | |If the subtracted has a greater name than the minuend, as if you subtract fourths from seconds, if they are fractions, the result are seconds of integers, and if they are integers, the result are seconds of fractions. |
| − | |style="text-align:right;"|ואם הנגרע גדול השם מאשר גרעון ממנו כגון שתגרע רביעיי' משניי' אם הם בשברי' יצאו שניי' שלמי‫' | + | :<math>\scriptstyle{\color{blue}{10^{2-4}=\frac{1}{10^2}\quad\frac{1}{10^{2-4}}=10^2}}</math> |
| + | |style="text-align:right;"|ואם הנגרע גדול השם מאשר גרעון ממנו כגון שתגרע רביעיי' משניי' אם הם בשברי' יצאו שניי' שלמי' ואם הם בשלמי' יצאו שברי' שניים | ||
| + | |- | ||
| + | |When you multiply integers by integers or fractions by fractions: | ||
| + | |style="text-align:right;"|וכשתכפל שלמי' בשלמי' או שברי' בשברי‫' | ||
| + | |- | ||
| + | |Add the names of the ranks [one to the other] and the product is [in the rank whose name is their sum]. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{10^n\sdot10^m=10^{n+m}}}</math> | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\frac{1}{10^n}\sdot\frac{1}{10^m}=\frac{1}{10^{n+m}}}}</math> | ||
| + | |style="text-align:right;"|חבר שם המדרגו' ושם יפול הנכפל | ||
| + | |- | ||
| + | |When you multiply integers by fractions: | ||
| + | |style="text-align:right;"|וכשתכפל שלמי' בשברי‫' | ||
|- | |- | ||
| − | | | + | |If their names are the same, the product is in the rank of units. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{OliveGreen}{10^n\sdot\frac{1}{10^n}=10^0}}</math> |
| + | |style="text-align:right;"|אם הם שוים בשם יפול הנכפל באחדי‫' | ||
|- | |- | ||
| − | | | + | |If not, subtract the names of the smaller from the name of the greater and the product is as the name that remains after the subtraction. |
| + | :<math>\scriptstyle{\color{OliveGreen}{m<n\;10^n\sdot\frac{1}{10^m}=10^{n-m}}}</math> | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{m>n\;10^n\sdot\frac{1}{10^m}=\frac{1}{10^{m-n}}}}</math> | ||
|style="text-align:right;"|ואם לאו גרע שם הקטן מן שם הגדול ושם יפול הנכפל [..] בצד הגדול בשם הנשאר אחר הגרעון | |style="text-align:right;"|ואם לאו גרע שם הקטן מן שם הגדול ושם יפול הנכפל [..] בצד הגדול בשם הנשאר אחר הגרעון | ||
|- | |- | ||
| − | | | + | |As if you multiply 3 seconds of integers by 2 sevenths of fractions: subtract 2 from 7; the result is 5. We find that the result of the multiplication is 6 fifths of fractions. |
| + | :<math>\scriptstyle{\color{blue}{\left(3\sdot10^{2}\right)\times\left(2\sdot\frac{1}{10^7}\right)=6\sdot\frac{1}{10^{7-2}}=6\sdot\frac{1}{10^{5}}}}</math> | ||
|style="text-align:right;"|כאלו תכפול ג' שלמי' שניי' בב' שברי' שביעיי' תגרע ב' מז' וישאר ה נמצ' יעלה הכפל ו' שברי' חמשיים | |style="text-align:right;"|כאלו תכפול ג' שלמי' שניי' בב' שברי' שביעיי' תגרע ב' מז' וישאר ה נמצ' יעלה הכפל ו' שברי' חמשיים | ||
|- | |- | ||
| − | | | + | |If 3 seconds of fractions by 2 sevenths of integers, the product is 6 fifths of integers. |
| + | :<math>\scriptstyle{\color{blue}{\left(3\sdot\frac{1}{10^2}\right)\times\left(2\sdot10^{7}\right)=6\sdot10^{7-2}=6\sdot10^{5}}}</math> | ||
|style="text-align:right;"|ואם ג' שברי' שניי' בב' שלמי' שביעיי' יהיה הנכפל ו' שלמי' חמשיים | |style="text-align:right;"|ואם ג' שברי' שניי' בב' שלמי' שביעיי' יהיה הנכפל ו' שלמי' חמשיים | ||
|- | |- | ||
| − | | | + | |This is the rule: when you divide a number by a number and they are both integers or both fractions: |
| − | |style="text-align:right;"|וזו לך צורה לזה כשתחלק מספר על מספר ושניהם שלמי' או שניה' שברי' אם שם מדרגותיה' שוה תפול החלוק באחדי' לעולם | + | |style="text-align:right;"|וזו לך צורה לזה כשתחלק מספר על מספר ושניהם שלמי' או שניה' שברי‫' |
| + | |- | ||
| + | |If the names of their ranks are the same, the quotient is always in the rank of units. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{10^n\div10^n=10^{n-n}=10^0}}</math> | ||
| + | |style="text-align:right;"|אם שם מדרגותיה' שוה תפול החלוק באחדי' לעולם | ||
|- | |- | ||
| − | | | + | |If the name of the upper is greater than [the name of] the lower, subtract the name of the lower from [the name of the upper] and the quotient is as [the name] that remains [of the same type], i.e. integers, if [both are] integers, or fractions, if [both are] fractions. |
| + | :<math>\scriptstyle{\color{OliveGreen}{m<n\;10^n\div10^m=10^{n-m}}}</math> | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{m<n\;\frac{1}{10^n}\div\frac{1}{10^m}=\frac{1}{10^{n-m}}}}</math> | ||
|style="text-align:right;"|ואם שם העליון גדול מהתחתון גרע שם התחתון ממנו וכמספר הנשאר תפול החלוקה בצד ההו' ר"ל אם הם שלמי' שלמי' ואם שברי' שברי‫' | |style="text-align:right;"|ואם שם העליון גדול מהתחתון גרע שם התחתון ממנו וכמספר הנשאר תפול החלוקה בצד ההו' ר"ל אם הם שלמי' שלמי' ואם שברי' שברי‫' | ||
|- | |- | ||
| − | | | + | |If the name of the lower is greater, subtract [the name of] the greater from [the name of the lower] and the quotient is as [the name] that remains of the opposite type i.e.: fractions, if both the divisor and the dividend are integers, or integers, if both are fractions. |
| + | :<math>\scriptstyle{\color{OliveGreen}{n<m\;10^n\div10^m=\frac{1}{10^{m-n}}}}</math> | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{n<m\;\frac{1}{10^n}\div\frac{1}{10^m}=10^{m-n}}}</math> | ||
|style="text-align:right;"|ואם שם התחתון גדול גרע העליון ממנו וכמספר הנשאר תפול החלוק בהפך הצד ר"ל בשברי' אם החולק והנחלק שלמי' ובשלמי' אם הם שברים | |style="text-align:right;"|ואם שם התחתון גדול גרע העליון ממנו וכמספר הנשאר תפול החלוק בהפך הצד ר"ל בשברי' אם החולק והנחלק שלמי' ובשלמי' אם הם שברים | ||
|- | |- | ||
| − | | | + | |If one is integer and the other is fraction and the names of their ranks are the same, or not the same, add the names of their ranks and the quotient is as the name of the sum, of the type of the upper number, i.e. the dividend: integer, if [the upper] is an integer, or fraction, if [the upper is] a fraction,. |
| + | :<math>\scriptstyle{\color{OliveGreen}{\frac{1}{10^n}\div10^m=\frac{1}{10^{n+m}}}}</math> | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{10^n\div\frac{1}{10^m}=10^{n+m}}}</math> | ||
|style="text-align:right;"|ואם האחד שלם והשני שברי' יהיה שם מדרגותיהם שוה או בלתי שוה חבר שמות המדרגו' יחד וכמספר המחובר יהיה שם הנופל בחלוק לצד שבו היה המספר העליון ר"ל המתחלק אם שלמי' בשלמי' ושברי' בשברי‫' | |style="text-align:right;"|ואם האחד שלם והשני שברי' יהיה שם מדרגותיהם שוה או בלתי שוה חבר שמות המדרגו' יחד וכמספר המחובר יהיה שם הנופל בחלוק לצד שבו היה המספר העליון ר"ל המתחלק אם שלמי' בשלמי' ושברי' בשברי‫' | ||
|- | |- | ||
| − | |||
| | | | ||
| − | + | ||
| + | === MS Paris 1081 15r-16v === | ||
| + | |||
| | | | ||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |Said Immanuel the son of Jacob: Since the division procedure is more difficult for the students than the multiplication procedure, and this is the way for the wise arithmeticians to extract the unknown number from the knowledge of the remaining five numbers and this is done by two multiplications and two divisions in different ways, we found it appropriate to explain how to extract the unknown from the knowledge of the remaining five and this is done by three multiplications and one division and I first give some signs for this procedure: |
| − | + | |style="text-align:right;"|‫<ref>15r</ref>אמר עמנואל בן יעקב לפי שמעשה החלוק יותר קשה למתלמדים ממעשה הכפל והיא הדרך לשאר החשבנים החכמים להוציא המספר האחד הנעלם איזה שיהיה מתוך ידיעת החמשה מספרים הנשארים ויעשה בזה שני כפלים ושני חלקים בדרכים שונים ראינו לבאר להוציא הנעלם מתוך ידיעת החמשה הנשארים ויעשה בזה שלשה כפלים וחלוק אחד ואומר ראשונה לתת קצת אותות אל זה המעשה | |
| − | |||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |Let the ratio of A to B consist of the ratio of G to D and the ratio of H to W. |
| − | { | + | :<math>\scriptstyle{\color{blue}{A:B=\left(G:D\right)\sdot\left(H:W\right)}}</math> |
| + | |style="text-align:right;"|הנה יחס א' אל ב' מחובר מיחס ג' אל ד' ומיחס ה' אל ו‫' | ||
|- | |- | ||
| − | | | + | |It has already been explained in [V].23 of Euclid that the ratio of the product of G by H to the product of D by W consists of the ratio of side G to side D and the ratio of side H to side W. |
| + | :<math>\scriptstyle{\color{blue}{\left(G\sdot H\right):\left(D\sdot W\right)=\left(G:D\right)\sdot\left(H:W\right)}}</math> | ||
| + | |style="text-align:right;"|וכבר התבאר מתמונת כ"ג מששי אקלידיס שיחס השטח ההווה מן ג' בה' אל השטח ההוה מן ד' בו' מחובר מיחס צלע ג' אל צלע ד' ומיחס צלע ה' אל צלע ו‫' | ||
|- | |- | ||
| − | | | + | |So, the ratio of A to B is the same as the ratio of the product of G by H to the product of D by W. |
| + | :<math>\scriptstyle{\color{blue}{A:B=\left(G\sdot H\right):\left(D\sdot W\right)}}</math> | ||
| + | |style="text-align:right;"|אם כן יחס [א]' אל ב' כיחס השטח ההווה מן ג' בה' אל השטח ההווה מן ד' בו‫' | ||
|- | |- | ||
| − | | | + | |Let the product of G by H be the number Z and the product of D by W be the number T. |
| + | :<math>\scriptstyle{\color{blue}{G\sdot H=Z\quad D\sdot W=T}}</math> | ||
| + | |style="text-align:right;"|ויהיה שטח ג' בה' מספר ז' ושטח ד' בו' מספר ט‫' | ||
|- | |- | ||
| − | | | + | |Hence, the ratio of A to B is the same as the ratio of Z to T. |
| + | :<math>\scriptstyle{\color{blue}{A:B=Z:T}}</math> | ||
| + | |style="text-align:right;"|אם כן יחס א' אל ב' כיחס ז' אל ט‫' | ||
|- | |- | ||
| − | | | + | |We multiply the extremes, which are A by T; let it be L. |
| + | :<math>\scriptstyle{\color{blue}{A\sdot T=L}}</math> | ||
| + | |style="text-align:right;"|אם כן נכפול הקצות שהם א' בט' ויהיה ל‫' | ||
|- | |- | ||
| − | | | + | |We divide L by the mean, which is B; the result is Z, which is the other mean. |
| + | :<math>\scriptstyle{\color{blue}{\frac{L}{B}=Z}}</math> | ||
| + | |style="text-align:right;"|ונחלק ל' על האמצעי והוא ב' ויעלה הז' שהוא האמצעי האחר | ||
|- | |- | ||
| − | | | + | |Or, we divide L by the mean, which is Z; the result is B, which is the other mean. |
| − | + | :<math>\scriptstyle{\color{blue}{\frac{L}{Z}=B}}</math> | |
| + | |style="text-align:right;"|או נחלק ל' על האמצעי והוא ז' ויעלה ב' והוא האמצע האחר | ||
|- | |- | ||
| − | | | + | |Or, we multiply the means, which are B by Z; it is L. We divide it by one extreme, which is T; the result is A, which is the other extreme. |
| − | + | :<math>\scriptstyle{\color{blue}{\frac{B\sdot Z}{T}=\frac{L}{T}=A}}</math> | |
| − | + | |style="text-align:right;"|או נכפול האמצעיים שהם ב' בז' ויהיה ל' ונחלק על הקצה ‫<ref>15v</ref>האחד והוא ט' ויעלה א' שהוא הקצה האחר | |
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |This is clear. |
| − | + | |style="text-align:right;"|וזה מבואר | |
|- | |- | ||
| − | | | + | |Thus, we explained [the case] when the first, which is A, is unknown, or the second, which is B, is unknown. |
| + | |style="text-align:right;"|ובזה ביארנו אם הראשון והוא א' נעלם <s>והו</s> או השני והוא ב' נעלם | ||
|- | |- | ||
| − | | | + | |It is explained in the eighteen propositions in the Conic Section of Menelaus that when the ratio of the first, which is A, to B, which is the second, consists of the ratio of G, which is the third, to D, which is the fourth, and the ratio of H, which is the fifth, to W, which is the sixth, then also the ratio of the third, which is G, to the fourth, which is D, consists of the ratio of the first, which is A to the second, which is B, and the ratio of the sixth, which is W, to the fifth, which is H. This is the eleventh proposition in the mentioned book. |
| + | :<math>\scriptstyle{\color{blue}{A:B=\left(G:D\right)\sdot\left(H:W\right)}}</math> | ||
| + | :<math>\scriptstyle{\color{blue}{G:D=\left(A:B\right)\sdot\left(W:H\right)}}</math> | ||
| + | |style="text-align:right;"|מבואר בתמונה החתוכית המיוחדת ל{{#annot:Menelaus|509|PoYr}}מילאוס{{#annotend:PoYr}} בשמנה עשר פנים שכאשר היה יחס הראשון והוא א' אל ב' והוא השני מחובר מיחס ג' והוא השלישי אל ד' והוא הרביעי ומיחס ה' אל ו' והוא החמשי אל ו' והוא הששי הנה גם כן יהיה יחס השלישי והוא ג' אל הרביעי והוא ד' מחובר מיחס הראשון והוא א' אל השני והוא ב' ומיחס הששי והוא ו' אל החמשי והוא ה' וזהו בפעם האחת עשרה עשרה מהתמונה הנזכרת | ||
|- | |- | ||
| − | | | + | |Therefore, we set the ratio as follows: third, first, fourth, second, first, third, second, fourth, sixth, fifth, fifth, sixth. |
| + | |style="text-align:right;"|אם כן נעשה היחס כן שלישי ראשון רביעי שני ראשון שלישי שני רביעי ששי חמשי חמשי ששי | ||
|- | |- | ||
| − | | | + | |Thus, according to the method we explained first, we can know [them] when the third is unknown, or when the fourth is unknown. |
| + | |style="text-align:right;"|אם כן על הדרך שביארנו ראשונה נוכל לדעת אם ג' שהיה שלישי נעלם או אם שהיה רביעי נעלם | ||
|- | |- | ||
| − | | | + | |It is also explained in the seventeenth proposition in the mentioned book that when the ratio of A, which is the first, to B, which is the second, consists of the ratio of G, which is the third, to D, which is the fourth, and the ratio of H, which is the fifth, to W, which is the sixth, then also the ratio of the fifth, which is H, to the sixth, which is W, consists of the ratio of the first, which is A to the second, which is B, and the ratio of the fourth, which is D, to the third, which is G. |
| + | :<math>\scriptstyle{\color{blue}{A:B=\left(G:D\right)\sdot\left(H:W\right)}}</math> | ||
| + | :<math>\scriptstyle{\color{blue}{H:W=\left(A:B\right)\sdot\left(D:G\right)}}</math> | ||
| + | |style="text-align:right;"|וגם כן יתבאר בפעם השבעה עשר מהתמונה הנזכרת שכאשר יחס א' והוא הראשון אל ב' והוא השני מחובר מיחס ג' והוא השלישי אל ד' והוא רביעיי ומיחס ה' והוא החמישי אל ו' והוא הששי הנה גם כן יהיה יחס החמשי והוא ה' אל הששי והוא ו' מחובר מיחס הראשון והוא א' <s>האמצעי</s> השני והוא ב' ומיחס הרביעי והוא ד' אל השלישי והוא‫<ref>16r</ref>ג‫' | ||
|- | |- | ||
| − | | | + | |Therefore, we set the ratio as follows: fifth, first, sixth, second, first, third, second, fourth, fifth, third, sixth. |
| + | |style="text-align:right;"|אם כן נעשה היחס כן חמשי ראשון ששי שני ראשון שלישי שני רביעי חמשי שלישי ששי | ||
|- | |- | ||
| − | | | + | |Thus, according to the method we explained first, we can know [them] when H, which is the fifth, is unknown, or when W, which is the sixth, is unknown. |
| − | + | |style="text-align:right;"|אם כן על הדרך שביארנו ראשונה נוכל לדעת אם ה' שהיה חמשי נעלם או ו' שהוא ששי נעלם | |
|- | |- | ||
| − | | | + | |The educated will understand. |
| − | + | |style="text-align:right;"|והמשכיל יבין | |
|- | |- | ||
| − | | | + | | colspan="2"| |
| − | + | {|class="wikitable" style="margin-left: auto; margin-right: auto; border: none; text-align:center;" | |
| − | |||
| − | |||
|- | |- | ||
| − | | | + | | || || ||ששי||חמשי||רביעי||שלישי||שני||ראשון |
| − | |||
| − | |||
| − | | | ||
|- | |- | ||
| − | | | + | |ל||ט‫'||ז‫'||ו‫'||ה‫'||ד‫'||ג‫'||ב‫'||א‫' |
| − | + | |} | |
| − | | | ||
|- | |- | ||
| − | | | + | | colspan="2"| |
| − | | | + | {|class="wikitable" style="margin-left: auto; margin-right: auto; border: none; text-align:center; color:blue" |
| − | {|class="wikitable" style="margin-left: auto; margin-right: | ||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | | || || ||sixth||fifth||fourth||third||second||first |
|- | |- | ||
| − | | | + | |L||T||Z||W||H||D||G||B||A |
|} | |} | ||
|- | |- | ||
| − | | | + | |If the first, which is A, is unknown: we multiply G by H; it is Z. We also multiply B by W; it is T. We also multiply B by Z; it is L. We divide L by T; the result is A, which is the unknown first. |
| − | {|style=" | + | :<math>\scriptstyle{\color{blue}{A=\frac{B\sdot\left(G\sdot H\right)}{B\sdot W}=\frac{B\sdot Z}{T}=\frac{L}{T}}}</math> |
| + | |style="text-align:right;"|אם הראשון והוא א' נעלם נכה ג' בה' והיה ז' עוד נכה ב' בו' והיה ט' עוד נכה ב' בז' והיה ל' ונחלק ל' על ט' ויצא א' שהוא הראשון הנעלם | ||
|- | |- | ||
| − | | | + | |If the [second], which is B, is unknown: we multiply G by H; it is Z. We also multiply B by W; it is T. We also multiply A by T; it is L. We divide L by Z; the result is B, which is the unknown second. |
| + | :<math>\scriptstyle{\color{blue}{B=\frac{A\sdot\left(B\sdot W\right)}{G\sdot H}=\frac{A\sdot T}{Z}=\frac{L}{Z}}}</math> | ||
| + | |style="text-align:right;"|אם הששי והוא ב' נעלם נכה ג' בה' והיה ז' עוד נכה ב' בו' והיה ט' עוד נכה א' בט' והיה ל' נחלק ל' על ז' ויצא ב' שהוא השני הנעלם | ||
|- | |- | ||
| − | | | + | |If the third, which is G, is unknown: we multiply A by W; it is Z. We also multiply B by H; it is T. We also multiply D by Z; it is L. We divide L by T; the result is G, which is the unknown third. |
| + | :<math>\scriptstyle{\color{blue}{G=\frac{D\sdot\left(A\sdot W\right)}{B\sdot H}=\frac{D\sdot Z}{T}=\frac{L}{T}}}</math> | ||
| + | |style="text-align:right;"|אם השלישי והוא ג' נעלם נכה א' בו' והיה ז' עוד נכה ב' בה' והיה ט' עוד נכה ד' בז' והיה ל' נחלק ל' על ט' ויצא ג' שהוא השלישי הנעלם | ||
|- | |- | ||
| − | | | + | |If the forth, which is D, is unknown: we multiply A by W; it is Z. We also multiply B by H; it is T. We also multiply G by T; it is L. We divide L by Z; the result is D, which is the unknown fourth. |
| − | | | + | :<math>\scriptstyle{\color{blue}{D=\frac{G\sdot\left(B\sdot H\right)}{A\sdot W}=\frac{G\sdot T}{Z}=\frac{L}{Z}}}</math> |
| + | |style="text-align:right;"|אם הרביעי והוא ד' נעלם נכה א' בו' והיה ז' עוד נכה ב' בה' והיה ט' עוד הכה ג' בט' והיה ל' נחלק ל' על ז' ויצא ד' שהוא הרביעי הנעלם | ||
|- | |- | ||
| − | | | + | |If the fifth, which is H, is unknown: we multiply A by D; it is Z. We also multiply B by G; it is T. We also multiply W by Z; it is L. We divide L by T; the result is H, which is the unknown fifth. |
| + | :<math>\scriptstyle{\color{blue}{H=\frac{W\sdot\left(A\sdot D\right)}{B\sdot G}=\frac{W\sdot Z}{T}=\frac{L}{T}}}</math> | ||
| + | |style="text-align:right;"|אם החמשי והוא ה' נעלם נכה א' בד' והיה ז' עוד נכה ב' בג' והיה ט' עוד נכה ו' בז' והיה ל' נחלק ל' על ט' ויצא ה' שהוא החמשי הנעלם | ||
|- | |- | ||
| − | | | + | |If the sixth, which is W, is unknown: we multiply A by D; it is Z. We also multiply B by G; it is T. We also multiply H by T; it is L. We divide L by Z; the result is W, which is the unknown sixth. |
| − | {|style=" | + | :<math>\scriptstyle{\color{blue}{W=\frac{H\sdot\left(B\sdot G\right)}{A\sdot D}=\frac{H\sdot T}{Z}=\frac{L}{Z}}}</math> |
| + | |style="text-align:right;"|אם הששי והוא ו' נעלם נכה א' בד' והיה ז' עוד נכה ב' בג' והיה ט' עוד נכה ה' בט' והיה ל' נחלק ל' על ז' ויצא ו' שהוא הששי הנעלם | ||
|- | |- | ||
| − | | | + | |This is about the way I explained to extract the unknown from the six by the knowledge of the remaining five. Understand [this]. |
| − | + | |style="text-align:right;"|זהו על דרך שבארתי להוציא הנעלם איזה שיהיה מהששה מתוך ידיעת החמשה הנשארים ובין | |
|- | |- | ||
| − | | | + | | |
| + | |||
| + | == Table – multiplication of sexagesimal fractions (Firenze) == | ||
| + | |style="text-align:right;"|<big>לוח כפילת המעלות ושבריהם</big> | ||
|- | |- | ||
| − | | | + | | colspan="2"| |
| − | + | {|class="wikitable" style="margin-left: auto; margin-right: auto; border: none; text-align:center;" | |
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |חמישיים||רביעיים||שלישיים||שניים||שברים||מעלות|| |
| − | |||
| − | | | ||
|- | |- | ||
| − | | | + | |חמישיים||רביעיים||שלישיים||שניים||שברים||מעלות||מעלות |
| − | |||
| − | |||
| − | | | ||
|- | |- | ||
| − | | | + | |ששיים||חמישיים||רביעיים||שלישיים||שניים||שברים||שברים |
| − | |||
| − | |||
| − | | | ||
|- | |- | ||
| − | | | + | |שבעיים||ששיים||חמישיים||רביעיים||שלישיים||שניים||שניים |
| − | |||
| − | | | ||
|- | |- | ||
| − | | | + | |שמיניים||שביעיים||ששיים||חמישיים||רביעיים||שלישיים||שלישיים |
| − | |||
| − | |||
| − | | | ||
|- | |- | ||
| − | | | + | |תשיעיים||שמיניים||שביעיים||ששיים||חמישיים||רביעיים||רביעיים |
| − | |||
| − | |||
| − | | | ||
|- | |- | ||
| − | | | + | |עשיריים||תשיעיים||שמיניים||שביעיים||ששיים||חמשיים||חמישיים |
| − | + | |} | |
| − | |||
|- | |- | ||
| − | | | + | | colspan="2"| |
| − | + | {|class="wikitable" style="margin-left: auto; margin-right: auto; border: none; text-align:center; color:blue" | |
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |fifths||fourths||thirds||seconds||primes||degrees|| |
| − | |||
| − | | | ||
|- | |- | ||
| − | | | + | |fifths||fourths||thirds||seconds||primes||degrees||degrees |
| − | |||
| − | |||
| − | | | ||
|- | |- | ||
| − | | | + | |sixths||fifths||fourths||thirds||seconds||primes||primes |
| − | |||
| − | |||
| − | | | ||
|- | |- | ||
| − | | | + | |sevenths||sixths||fifths||fourths||thirds||seconds||seconds |
| − | |||
| − | | | ||
|- | |- | ||
| − | | | + | |eighths||sevenths||sixths||fifths||fourths||thirds||thirds |
| − | |||
| − | |||
| − | | | ||
|- | |- | ||
| − | | | + | |ninths||eighths||sevenths||sixths||fifths||fourths||fourths |
| − | |||
| − | |||
| − | | | ||
|- | |- | ||
| − | | | + | |tenths||ninths||eighths||sevenths||sixths||fifths||fifths |
| − | + | |} | |
| − | |||
| − | | | ||
| − | |||
|- | |- | ||
| | | | ||
| − | + | ||
| − | + | == Table – multiplication of sexagesimal fractions (Firenze) == | |
| − | |style="text-align:right;"| | + | |
| + | |style="text-align:right;"|<big>לוח חילוק השברים האחד על השני</big> | ||
|- | |- | ||
| − | | | + | | colspan="2"| |
| − | + | {|class="wikitable" style="margin-left: auto; margin-right: auto; border: none; text-align:center;" | |
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |ששיים||חמישיים||רביעיים||שלישיים||שניים||שברים||מעלות |
| − | |||
| − | | | ||
|- | |- | ||
| − | | | + | |חמישיים||רביעיים||שלישיים||שניים||שברים||מעלות||שברים |
| − | | | ||
|- | |- | ||
| − | | | + | |רביעיים||שלישיים||שניים||שברים||מעלות||שברים||שניים |
| − | | | + | |- |
| + | |שלישיים||שניים||שברים||מעלות||שברים||שניים||שלישיים | ||
| + | |- | ||
| + | |שניים||שברים||מעלות||שברים||שניים||שלישיים||רביעיים | ||
| + | |- | ||
| + | |שברים||מעלות||שברים||שניים||שלישיים||רביעיים||חמישיים | ||
|- | |- | ||
| − | | | + | |מעלות||שברים||שניים||שלישיים||רביעיים||חמישיים||ששיים |
| − | | | + | |} |
|- | |- | ||
| − | | | + | | colspan="2"| |
| − | + | {|class="wikitable" style="margin-left: auto; margin-right: auto; border: none; text-align:center; color:blue" | |
|- | |- | ||
| − | | | + | |sixths||fifths||fourths||thirds||seconds||primes||degrees |
| − | | | ||
|- | |- | ||
| − | | | + | |fifths||fourths||thirds||seconds||primes||degrees||primes |
| − | | | ||
| − | |||
|- | |- | ||
| − | | | + | |fourths||thirds||seconds||primes||degrees||primes||seconds |
| − | | | ||
| − | |||
|- | |- | ||
| − | | | + | |thirds||seconds||primes||degrees||primes||seconds||thirds |
|- | |- | ||
| − | | | + | |seconds||primes||degrees||primes||seconds||thirds||fourths |
| − | | | ||
|- | |- | ||
| − | | | + | |primes||degrees||primes||seconds||thirds||fourths||fifths |
|- | |- | ||
| + | |degrees||primes||seconds||thirds||fourths||fifths||sixths | ||
|} | |} | ||
| − | |||
|- | |- | ||
| | | | ||
| − | + | ||
| − | + | == Multiplication of integers (Firenze) == | |
| | | | ||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *Example for the multiplication methods: we wish to multiply 127 by 355. |
| + | :<math>\scriptstyle127\times355</math> | ||
| + | |style="text-align:right;"|דמיון על דרכי הכפל רצינו לכפול קכ"ז על שנ"ה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::We write 127 on the upper line and 355 on the other lower [line]. This is its diagram: |
| + | |style="width:45%; text-align:right;"|וכתבנו בטור העליון קכ"ז ובשני השפל שנ"ה וזה צורתו | ||
|- | |- | ||
| | | | ||
| − | |style="text-align: | + | ::{|class="wikitable" style="color:blue; text-align:center; |
|- | |- | ||
| | | | ||
| − | |style=" | + | {|style="margin-left: auto; margin-right: 0px;" |
|- | |- | ||
| − | | | + | |1||2||7 |
| − | | | ||
|- | |- | ||
| + | |3||5||5 | ||
|} | |} | ||
| − | |||
|- | |- | ||
| | | | ||
| − | + | {|style="margin-left: auto; margin-right: 0px;" | |
| − | + | |- | |
| − | | | + | | ||2||4||8||5 |
| + | |- | ||
| + | | ||7||1||0|| | ||
|- | |- | ||
| − | | | + | |3||5||5|| || |
| − | | | + | |} |
|- | |- | ||
| − | | | + | |the sum |
| − | |||
|- | |- | ||
| | | | ||
| − | |style=" | + | {|style="margin-left: auto; margin-right: 0px;" |
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |4||5||0||8||5 |
| − | | | + | |} |
|- | |- | ||
| + | |} | ||
| | | | ||
| − | |style="text-align: | + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" |
|- | |- | ||
| | | | ||
| − | |style=" | + | {|style="margin-left: auto; margin-right: 0px;" |
|- | |- | ||
| − | | | + | |א||ב||ז |
| − | | | ||
|- | |- | ||
| − | | | + | |ג||ה||ה |
| − | + | |} | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | | | ||
| − | | | ||
| − | | | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | | | ||
| − | |||
|- | |- | ||
| | | | ||
{|style="margin-left: auto; margin-right: 0px;" | {|style="margin-left: auto; margin-right: 0px;" | ||
|- | |- | ||
| − | | | + | | ||ב||ד||ח||ה |
|- | |- | ||
| − | | || | + | | ||ז||א||0|| |
|- | |- | ||
| − | | || | + | |ג||ה||ה|| || |
|} | |} | ||
|- | |- | ||
| − | | | + | |המחובר |
|- | |- | ||
| | | | ||
| − | |style=" | + | {|style="margin-left: auto; margin-right: 0px;" |
| − | + | |- | |
| − | + | |ד||ה||0||ח||ה | |
| − | + | |} | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | ד | ||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |} |
| − | + | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::We multiply 7 by 5; it is 35. |
| − | + | ::<math>\scriptstyle{\color{blue}{7\times5=35}}</math> | |
| − | + | |style="text-align:right;"|כפלנו ז' על ה' והוא ל"ה | |
| − | + | |- | |
| + | | | ||
| + | ::We write 5 in the first rank, corresponding to 7; and keep the 3, in order to add it to the second rank. | ||
| + | |style="text-align:right;"|כתבנו ה' במעלה הראשונה כנגד ז' ושמרנו הג' כדי לצרף אותה אל המעלה השנייה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::We multiply 7 by the second lower 5; the result is 35. |
| − | + | ::<math>\scriptstyle{\color{blue}{7\times5=35}}</math> | |
| + | |style="text-align:right;"|עוד כפלנו ז' על ה' השני התחתון עלו ל"ה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::We add the 5 to the 3 we kept; the result is 8. |
| + | ::<math>\scriptstyle{\color{blue}{5+3=8}}</math> | ||
| + | |style="text-align:right;"|חברנו הה' עם הג' ששמרנו ועלו ח‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::We write 8 in the second rank, beneath the 2 of the upper line and we write the 3 in the third rank, beneath the 1 of the upper line, with the result of multiplication of that rank. |
| + | |style="text-align:right;"|ושמנו ח' במעלה השנית תחת ב' מטור העליון והג' שמנו במעלה השלישית תחת הא' מטור העליון עם היוצא מכפל אותה המעלה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::We multiply the first 7 by the lower 3; the result is 21. |
| − | + | ::<math>\scriptstyle{\color{blue}{7\times3=21}}</math> | |
| − | + | |style="text-align:right;"|עוד כפלנו ז' הראשון על הג' התחתון עלו כ"א | |
| − | + | |- | |
| − | + | | | |
| − | + | ::We add the 1 to the 3 we kept; it is 84. | |
| − | + | ::<math>\scriptstyle{\color{blue}{1+3=4}}</math> | |
| − | + | |style="text-align:right;"|חברנו א' עם הג' ששמרנו והיו ד‫' | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| − | |||
| | | | ||
| − | + | ::We write 4 beneath the 1 in the third rank and 2 in the fourth rank. | |
| + | |style="text-align:right;"|וכתבנו ד' תחת הא' במעלה השלישית וב' במעלה רביעית | ||
|- | |- | ||
| − | |||
| | | | ||
| − | {|style=" | + | ::We multiply the upper middle 2 by the first 5 on the lower line; the result is ten. |
| + | ::<math>\scriptstyle{\color{blue}{2\times5=10}}</math> | ||
| + | |style="text-align:right;"|עוד כפלנו ב' האמצעי העליון על ה' הראשון מן הטור השפל עלו עשר | ||
|- | |- | ||
| − | | | + | | |
| − | + | ::We write a zero in the second [rank] and 1 in the third [rank], beneath the 3 of the lower line. | |
| + | |style="text-align:right;"|כתבנו ציפרא בשינית וא' בשלישית תחת הג' שבטור השפל | ||
|- | |- | ||
| − | |||
| | | | ||
| − | {|style=" | + | ::We multiply the upper 2 by the second upper 5; the result is ten. |
| + | ::<math>\scriptstyle{\color{blue}{2\times5=10}}</math> | ||
| + | |style="text-align:right;"|עוד כפלנו ב' העליון על הה' השני התחתון עלו עשר | ||
|- | |- | ||
| − | | | + | | |
| − | + | ::We add the 10 to the 1 we kept; it is 11. | |
| + | ::<math>\scriptstyle{\color{blue}{10+1=11}}</math> | ||
| + | |style="text-align:right;"|חברנו י' עם הא' ששמרנו והיא י"א | ||
|- | |- | ||
| − | |||
| | | | ||
| − | + | ::We write 1 in the third [rank] and keep the 1 in the fourth [rank]. | |
| + | |style="text-align:right;"|שמנו א' בשלישית וא' שמרנו ברביעית | ||
|- | |- | ||
| − | | | + | | |
| + | ::We multiply 2 by 3; it is six. | ||
| + | ::<math>\scriptstyle{\color{blue}{2\times3=6}}</math> | ||
| + | |style="text-align:right;"|עוד כפלנו ב' על ג' והיו ששה | ||
|- | |- | ||
| − | | | + | | |
| + | ::We add the 6 to the 1; it is 7. We write it in the fourth [rank]. | ||
| + | ::<math>\scriptstyle{\color{blue}{6+1=7}}</math> | ||
| + | |style="text-align:right;"|חברנו הו' עם הא' והיו ז' וכתבנוהו ברביעית | ||
|- | |- | ||
| − | |||
| − | |||
| | | | ||
| − | {|style=" | + | ::We multiply 1 by the first 5 in the lower line; we write 5 in the third [rank]. |
| + | ::<math>\scriptstyle{\color{blue}{1\times5=5}}</math> | ||
| + | |style="text-align:right;"|עוד כפלנו א' על ה' הראשונ שבטור השפל וכתבנו ה' בשלישית<br> | ||
| + | עוד כפלנו א' על ה' הראשון שבטור השפל וכתבנו ה' בשלישית | ||
|- | |- | ||
| − | | | + | | |
| + | ::We multiply 1 by the second 5; we write 5 in the fourth [rank]. | ||
| + | ::<math>\scriptstyle{\color{blue}{1\times5=5}}</math> | ||
| + | |style="text-align:right;"|עוד כפלנו א' על ה' השני וכתבנו ה' ברביעית | ||
|- | |- | ||
| − | | | + | | |
| + | ::We multiply 1 by 3; we write 3 in the fifth [rank]. | ||
| + | ::<math>\scriptstyle{\color{blue}{1\times3=3}}</math> | ||
| + | |style="text-align:right;"|עוד כפלנו א' על ג' וכתבנו ג' במעלה החמישית | ||
|- | |- | ||
| − | | | + | | |
| − | | | + | ::The multiplication is complete. |
| + | |style="text-align:right;"|נשלם כפל החבור | ||
|- | |- | ||
| | | | ||
| − | + | ::We sum up the result of multiplication of the two rows: | |
| + | |style="text-align:right;"|נבוא לחבר היוצא מכפל שני הטורים | ||
|- | |- | ||
| − | | | + | | |
| + | ::We write 5 in the first rank. | ||
| + | |style="text-align:right;"|ונכתוב ה' לכח במעלה הראשונה | ||
|- | |- | ||
| − | | | + | | |
| + | ::8 in the second. | ||
| + | |style="text-align:right;"|וח' בשנית | ||
|- | |- | ||
| − | |||
| − | |||
| | | | ||
| − | {|style=" | + | ::4, 1, 5 are ten; we write 0 in the third and mark a point in the fourth to sign the ten. |
| + | ::<math>\scriptstyle{\color{blue}{4+1+5=10}}</math> | ||
| + | |style="text-align:right;"|וד' וא' וה' עולים עשרה נעשה 0' בשלישית ונשים נקודה ברביעית להיות לזיכר בעבו' העשרה | ||
|- | |- | ||
| − | | | + | | |
| + | ::We sum up 2, 7, 5; it is 14. Then the reserved; it is 15. We write 5 in the fourth rank and a point in the fifth. | ||
| + | ::<math>\scriptstyle{\color{blue}{5+7+2+1=14+1=15}}</math> | ||
| + | |style="text-align:right;"|ואחר נחבר ב'ז'ה' והם י"ד ואחר השמור הרי ט"ו נשים ה' במעלה הרביעית ונקודה בחמישית | ||
|- | |- | ||
| − | | | + | | |
| − | |- | + | ::We add one to the 3; it is 4. We write 4 in the fifth rank. |
| − | + | ::<math>\scriptstyle{\color{blue}{1+3=4}}</math> | |
| − | + | |style="text-align:right;"|נחבר אחד עם הג' ויהיו ד' ונכתוב ד' במעלה החמשית | |
|- | |- | ||
| − | |||
| | | | ||
| − | + | ::The addition is complete. | |
| + | |style="text-align:right;"|נשלם החבור | ||
|- | |- | ||
| − | | | + | |The scales of multiplication by [casting out] the nines |
| − | + | |style="text-align:right;"|<big>המאזניים מן הכפל על חש' ט' ט‫'</big> | |
|- | |- | ||
| − | | | + | | |
| + | ::We consider the [digits] in the upper row as if they were units; they are ten according to this way. | ||
| + | |style="text-align:right;"|הנה חשבנו חשבון הטור העליון כאילו הם אחדים והיו עשרה כזה הדרך | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | ::We cast out the nine; 1 remains. We write it next to the upper row. |
| − | + | ::<math>\scriptstyle{\color{blue}{10-9=1}}</math> | |
| − | + | |style="text-align:right;"|השלכנו התשעה ונשאר א' וכתבנוהו בצד הטור העליון | |
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | + | ::{|class="wikitable" style="color:blue; text-align:center; | |
| − | == | + | |- |
| − | | | + | |1 |
| + | |rowspan="2"| | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |1||2||7 | ||
|- | |- | ||
| − | | | + | |3||5||5 |
| − | | | + | |} |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |4 |
| − | |||
|- | |- | ||
| − | | | + | |} |
| − | + | | | |
| − | + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | |
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |א |
| − | + | |rowspan="2"| | |
| − | |style=" | + | {|style="margin-left: auto; margin-right: 0px;" |
| − | + | |- | |
| − | + | |א||ב||ז | |
| − | + | |- | |
| − | + | |ג||ה||ה | |
| − | + | |} | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |ד |
| − | |||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |} |
| − | + | ||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | ::Then, we calculate the bottom row; we find the result is 13. |
| − | + | |style="text-align:right;"|אחר כן חשבנו הטור השפל ומצאנוהו עולה י"ג | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | : | + | ::We subtract the 9; 4 remains. We write it next to the one. |
| − | :<math>\scriptstyle{\color{blue}{ | + | ::<math>\scriptstyle{\color{blue}{13-9=4}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|הפלנו הט' ונשארו ד' וכתבנום בצדו אחד |
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | ::Then we multiply 1 by 4; it is 4. We keep it. |
| − | :<math>\scriptstyle{\color{blue}{\ | + | ::<math>\scriptstyle{\color{blue}{1\times4=4}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואחר כפלנו א' על ד' והיה ד' ושמרנום |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | ::We calculate the result, which is 45085; the result is 22. |
| − | :<math>\scriptstyle{\color{blue}{ | + | ::<math>\scriptstyle{\color{blue}{4+5+0+8+5=22}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואחר חשבנו המחובר והיה זה ה ח 0 ה ד ועלה כ"ב |
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | ::We cast out 18, which is removed by nines; 4 remains and it is equal to the product of the scales of the two rows. Therefore we know that our calculation is correct. |
| − | :<math>\scriptstyle{\color{blue}{ | + | ::<math>\scriptstyle{\color{blue}{22-18=4}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|השלכנו י"ח שהולך לתשיעיות ונשארו ד' והוא שווה לכפל מאזני שני הטורים ואז ידענו שחשבונינו אמתי |
|- | |- | ||
| − | | | + | |The rule is that when one of the multiplicands of the product is cast out by nines, whether the upper or the bottom, then the result of the multiplication should be cast out by nines; otherwise not. |
| − | + | |style="text-align:right;"|והכלל הוא כי כשאחד ממדרגות הכפל הולך לתשיעיות הן העליון הן השפל היוצא מחבור הכפל ראוי ללכת בתשיעיות ואם לאו אינו צורך | |
| − | |||
| − | |style="text-align:right;"| | ||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |If both are not removed by nines, multiply the remainders of the two rows of multiplication by each other and see the remainder from nines, whether it is 5, or 6, or 7. |
| − | + | |style="text-align:right;"|ואם אינו הולך לתשיעיות כל אחד משניהם תכפול העודף משני טורי הכפל זה על זה וראה מה שיעדיף על תשיעיות אם ה' אם ו' אם ז‫' | |
| − | |||
| − | |style="text-align:right;"| | ||
|- | |- | ||
| − | | | + | |That is, see the result of the upper row and multiply it by the result of the bottom row. |
| − | + | |style="text-align:right;"|ר"ל תראה מה שיעלה הטור העליון ותכפלהו על מה שיעלה הטור השפל | |
| − | |||
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | : | + | ::As if there are 3 and 4 in the upper [row], then the result is 7; and in the bottom [row] there are 2 and 3, so the result is 5: multiply 7 by 5; the result is 35. The remainder from the nines you cast out is 8. |
| − | :<math>\scriptstyle{\color{blue}{\ | + | ::<math>\scriptstyle{\color{blue}{\left(3+4\right)\times\left(2+3\right)=7\times5=35_9\equiv 8}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כאלו יהיה בעליון ד' ג' שעולה ז' ובתחתון ג' ב' שעולה ה' תכפול ז' על ה' עולה ל"ה נשאר על תשיעיות שהשלכת ח‫' |
|- | |- | ||
| − | | | + | |The same will be in the product no more and no less, when you examine how much is the result of the multiplication and by how much it exceeds over nine. |
| − | |style="text-align:right;"|וכן | + | |style="text-align:right;"|וכן יהיה בחבור בלי פחות ויתר כאשר תעיין כמה חבור הכפל וכמה יעדיף על תשעה |
| − | |||
|- | |- | ||
| − | | | + | |If the total of the upper row and of the bottom row are less than 9, multiply the upper by the bottom, see by how much [the product] exceeds over nines and the same should be for the result of the multiplication, if the multiplication is correct; otherwise, it is incorrect. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואם בין הטור העליון והשפל לא יעלה הכל ט' תפיל העליון על השפל וראה מה שיעדיף על תשיעיות וככה יהיה החבור אם הכפל יצדק ואם לא יצדק תם |
| − | |||
|- | |- | ||
| | | | ||
| − | + | ||
| − | + | == Division of integers == | |
| − | |||
| − | |||
| − | |||
| | | | ||
| − | |||
|- | |- | ||
| − | | | + | |Conducted by Bonfils may his soul rest in paradise. |
| − | + | |style="text-align:right;"|‫<ref>Guenzburg 193v</ref>עשאו שין בונפייל נ"ע‫<ref>MS Firenze: ביאור החלוק עשאו ה'ה'ר' עמנואל בן יעקב ע"ב בעל הכנפיים; MS London: כלל שעשה החכם הגדול ר עמנואל בן יעקב למצוא דרך קל לכל חשבון קראו חכם אמר; MS P1081 אמר עמנואל בן יעקב</ref> | |
|- | |- | ||
| − | | | + | |When you want to divide many digits by many digits, however many there may be, write the number you wish to divide, which is the greater, in one row, each one according to its rank and call it "the upper row". |
| + | |style="text-align:right;"|<big>כשתרצה</big> לחלק מספרים רבים על מספרים רבים כמה שיהיו תשים המספר שתרצה לחלקו והוא ביותר גדול בטור כל אחד ואחד כפי מעלתו וקרא טור עליון‫<ref>על מספרים רבים כמה שיהיו: MS P1081 om.</ref> | ||
|- | |- | ||
| − | | | + | |Write the second number, by which you want to divide, and it is the smaller, in another row, beneath the upper row, each beneath its type, i.e.: units beneath units, tens beneath tens etc., and this row is called "the bottom row". |
| − | | | + | |style="text-align:right;"|והמספר השני שתרצה לחלק עליו והוא היותר קטן תשים בטור אחר תחת הטור העליון כל מין תחת מינו ר"ל אחדים תחת אחדים עשרות תחת עשרות וכו' שהטור יקרא הטור השפל‫<ref>בטור: MS L om.<br> |
| − | + | הטור: MS L om.<br> | |
| − | + | וכו‫': Lומאיות תחת מאיות<br> | |
| − | + | שהטור: MS L וזה<br> | |
| + | הטור השפל: MS L טור שפל</ref> | ||
|- | |- | ||
| − | | | + | |Leave a space between the two rows we mentioned, so that you can write the result of division between them and this row is called "the middle row". |
| − | + | |style="text-align:right;"|וריוח תשים בין שני הטורים שהזכרנו כדי שתוכל לכתוב ביניהם העולה בחלוק וזה הטור יקרא טור אמצעי‫<ref>הטור: MS L om.</ref> | |
|- | |- | ||
| − | | | + | |When you start dividing your number, consider all the digits as if they were units and see how many times the last digit of the bottom row is in the last digit of the upper row. |
| + | |style="text-align:right;"|וכאשר תחל לחלק חשבונך ותחשוב כל המספרי' כאלו הם אחדים תראה כמה פעמים יהיה המספר האחרון שבטור השפל כמספר אחרון שבטור עליון‫<ref>הם: Guenzburg: קח</ref> | ||
|- | |- | ||
| − | | | + | |In such way that the second to the last in the bottom row, i.e. before it, is the same number of times in the second to the last in the upper row before it. |
| − | + | |style="text-align:right;"|ובאופן שיהיה מספר הפעמים ההם השני לאחרון שבטור השפל ר"ל לפניו השני לאחרון שבטור העליון לפניו‫<ref>כמספר אחרון שבטור... לאחרון שבטור השפל: MS P1081 om.</ref> | |
|- | |- | ||
| − | | | + | |Also the third to the last in the bottom row is in the third to the last in the upper row and so on successively. |
| + | |style="text-align:right;"|וכן השלשי לאחרון שבטור <s>השל</s> השפל לפניו כשלשי לאחרון שבטור העליון לפניו וכן כולם כסדר הזה‫<ref>כשלשי לאחרון שבטור העליון לפניו: MS L om.</ref> | ||
|- | |- | ||
| − | | | + | |Write the number you receive with the previous method, i.e. the number of times the last digit in the bottom row is in the last digit in the upper row, in the middle row, i.e. between the upper row and the bottom row. Write this number as far from the last digit of the upper row, from which you take the quotient, as the [number of] ranks that the last digit of the bottom row is far from the rank of units. |
| − | + | |style="text-align:right;"|ואותו המספר אשר יעלה לך עם האופן הקודם ר"ל מספר הפעמים אשר יהיה המספר האחרון שבטור השפל כמספר האחרון שבטור העליון תכתוב בטור האמצעי ר"ל בין הטור העליון והשפל ותכתוב כמספר ההוא רחוק מהמספר האחרון שבטור העליון אשר תקח ממנו החלק כמו מדרגות אשר רחק כמספר האחרון שבטור השפל ממדרגת האחדים‫<ref>מספר: MS L כמה<br> | |
| − | |style="text-align:right;"| | + | הפעמים: MS L פעמים<br> |
| + | אשר: MS L om.<br> | ||
| + | האמצעי: MS L אמצע<br> | ||
| + | ותכתוב כמספר ההוא: MS L ותכתבינו<br> | ||
| + | תקח: MS L יקח<br> | ||
| + | שבטור: MS L om.<br> | ||
| + | ממדרגת: MS L שבמדרגת</ref> | ||
|- | |- | ||
| − | | | + | |Know that if you cannot take the last digit of the bottom row even once from the last digit of the upper row, as if we say for instance that the last of the bottom row is 1 and the last of the upper row is 8 or less, like our next example: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<big>ודע</big> לך שאם לא תוכל לקחת שום פעם המספר האחרון שבטור השפל כמספר האחרון שבטור העליון כאלו נאמר דרך משל שהאחרון שבטור השפל הוא א' והאחרון שבטור העליון הוא ח' או פחות כמו בדמיונו זה‫<ref>לך: MS L om.<br> |
| + | לא: MS L om.<br> | ||
| + | לקחת: MS L לדעת<br> | ||
| + | פעם: MS L om.<br> | ||
| + | המספר: MS L מספר<br> | ||
| + | האחרון: MS P1081 אחרון<br> | ||
| + | שבטור: MS P1081 שבטור שבטור<br> | ||
| + | הוא: MS L om.<br> | ||
| + | הוא א' ... העליון: MS P1081 om.<br> | ||
| + | הוא: MS L om.</ref> | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align: | + | ::{|class="wikitable" style="color:blue; text-align:center; |
|- | |- | ||
| − | | | + | | |
| − | |style=" | + | {|style="margin-left: auto; margin-right: 0px;" |
|- | |- | ||
| − | | | + | |8||9||4||3||2||1 |
| − | | | ||
|- | |- | ||
| − | | | + | | || || || ||9|| |
| − | | | ||
|- | |- | ||
| − | | | + | | || ||9||4||3||2 |
| − | | | + | |} |
| − | | | ||
| − | | | ||
| − | | | ||
| − | | | ||
| − | | | ||
| − | | | ||
|- | |- | ||
| + | |} | ||
| | | | ||
| − | + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | |
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
{|style="margin-left: auto; margin-right: 0px;" | {|style="margin-left: auto; margin-right: 0px;" | ||
|- | |- | ||
| − | | | + | |ח||ט||ד||ג||ב||א |
|- | |- | ||
| − | | | + | | || || || ||ט|| |
|- | |- | ||
| − | + | | || ||ט||ד||ג||ב | |
| + | |} | ||
|- | |- | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|} | |} | ||
|- | |- | ||
| | | | ||
| − | {|style=" | + | ::You shift the whole number backwards, i.e. the digit 8 to the 9 before it and take ten for each unit, i.e. with the 9 before the 8, they are 89. See how many times the 9, which is the last digit of the bottom row, is in 89. But, keep in mind that the digit 4, which is before the 9 in the bottom row, should be as many times in the digit 4, which is before the 9 in the upper row. As if you say that you can take 9 times 9, which is 81, from the number 89 and 8 remains. With the preceding 4, it is 84. So, you can take 9 times 4 from the number 84. |
| − | | | + | ::<math>\scriptstyle{\color{blue}{89-\left(9\times9\right)=89-81=8}}</math> |
| − | + | |style="text-align:right;"|אז תשוב כל המספר ההוא אחורנית ר"ל מספר <s>על</s> הח' על הט' שלפניו ב' ותקח לכל אחד עשרה ר"ל שעם הט' אשר לפני הח' יהיו פ"ט ותראה כמה פעמים יהיה ט' שהוא מספר האחרון שבטור השפל בפ"ט ותחשוב בעצמך שיהיה המספר ד' שהיא לפני הט' שבטור השפל בכמו הפעמים ההם באות הד' אשר לפני הט' שבטור העליון כאלו תאמר הנה תוכל לקחת ט' פעמים ט' <s>בכמו</s> במספר פ"ט שהם פ"א ונשארו שם ח' ועם הד' שלפניו יהיו פ"ד הנה אם כן תוכל לקחת ט' פעמים ד' במספר פ"ד‫<ref>כל המספר ... ר"ל: MS L om.<br> | |
| − | + | הח‫': MS L ח‫'<br> | |
| − | + | יהיו: MS L יהיה<br> | |
| − | + | ותראה: MS L וראה<br> | |
| − | + | יהיה: MS P1081 הוא<br> | |
| − | + | Guenzburg: בפניו<br> | |
| + | בעצמך: MS L כל כך<br> | ||
| + | המספר: MS L מספרו<br> | ||
| + | בפ"ט: Guenzburg om.<br> | ||
| + | שבטור העליון: MS L שבטור הח‫'<br> | ||
| + | ט‫': MS L om.<br> | ||
| + | ועם: MS L <s>חסרינו</s> עם<br> | ||
| + | הנה: MS L om.<br> | ||
| + | כן: MS L om.<br> | ||
| + | ד' במספר פ"ד: MS L om.</ref> | ||
|- | |- | ||
| | | | ||
| − | + | ::Therefore, take 9 and write it first in the middle row, as far from the digit, from which you took the quotient, which is 9, as the number [of ranks] that the last digit of the bottom row, which is 9, is far from the rank [of units], which is four ranks. So, write the 9 you receive from the division in the rank of tens, which is beneath the 2 of the upper row. | |
| + | |style="text-align:right;"|לזה תקח ט' ותכתבנה א' בטור האמצעי רחוק מהאות שלקחת החלוק ממנו שהוא ט' בכמו המספר אשר רחק האות האחרון שבטור השפל שהוא ט' ממדרגתו והם ארבע מדרגות לכן תכתוב בט' אשר יצא לך בחלוק במדרגת העשרות שהוא תחת הב' שבטור העליון‫<ref>ט‫': MS L ד' ט‫'<br> | ||
| + | ממדרגתו: MS L ממדרגות<br> | ||
| + | בט‫': MS P1081 הד‫'<br> | ||
| + | במדרגת: MS L במדרגות</ref> | ||
|- | |- | ||
| − | | | + | |Then, draw a line beneath the bottom row and multiply the digit you received in the division, i.e. 9 by the whole bottom row, according to the multiplication method you know. Subtract the product from the upper row, each from its type, starting from the rank nearest to the units, or from the units, if there are units. Write the remainder from subtraction above the upper row. |
| − | + | |style="text-align:right;"|אחר כן תשים קו תחת הטור השפל וכפול זאת האות שיצא לך בחלוק ר"ל ט' עם כל הטור השפל כאשר ידעת דרך הכפל והיוצא מהכפל גרע מהטור העליון כל מין ממינו ונתחיל מן המדרגה הקרובה אל האחדים או מן האחדים אם יש שם אחדים והנשאר אחר הגרעון‫<ref>Guenzburg 194r</ref> כתוב על הטור <s>הראשון</s> העליון‫<ref>כן: MS L om.<br> | |
| + | וכפול: MS L אחר זה כפול<br> | ||
| + | האות: Guenzburg om.<br> | ||
| + | זאת האות שיצא לך: MS L אשר יצא<br> | ||
| + | L: הכפל ר"ל ט' על ב' על ג' על ד' על ט‫'<br> | ||
| + | מהטור: MS L מטור<br> | ||
| + | או מן האחדים: MS L om.<br> | ||
| + | אחר: MS L מן<br> | ||
| + | הטור: MS L טור</ref> | ||
|- | |- | ||
| − | | | + | |After that, divide again what remains above the upper row by the bottom row in the manner we explained, then multiply the digit received from the division by the entire bottom row, as at the beginning, and subtract the product from what remains on the upper row and the remainder remains above it. |
| − | | | + | |style="text-align:right;"|אחר זה שוב לחלק הנשאר על הטור העליון על הטור השפל על הדרך שביארנו ואחר כפול האות היוצא בחלוק על כל הטור השפל כאשר בתחלה ואחר גרע העולה מהכפל מן הנשאר בטור העליון וישאר הנשאר עליו‫<ref>שוב: MS L ישוב<br> |
| − | + | על הדרך ... הטור השפל: MS L om.<br> | |
| + | מהכפל: MS L om.<br> | ||
| + | מן הנשאר: MS L מהנשאר</ref> | ||
|- | |- | ||
| − | | | + | |Divide it again, if there is something in it to divide, multiply and subtract as mentioned. |
| − | + | |style="text-align:right;"|ואחר תשוב לחלק עוד אם יש בה לחלק ולכפול ולגרוע כדבר האמור‫<ref>ואחר: MS L אחר כן<br> | |
| + | ולכפול: MS L לכפול</ref> | ||
|- | |- | ||
| − | |& | + | |The sign for this method is D.M.S. [in Hebrew ḥ.k.m.], i.e. division, multiplication, and subtraction. |
| + | |style="text-align:right;"|והסימן לזה הדרך ח'כ'ם' ר"ל חלוק כפל ומגרעת‫<ref>לזה הדרך: MS L om.</ref> | ||
|- | |- | ||
| − | | | + | |Always do this until what remains in the upper row is less than what is in the bottom row. |
| + | |style="text-align:right;"|וכן תעשה תמיד עד שישאר בטור<ref>בטור: MS P1081 הטור</ref> עליון פחות ממה שבטור<ref>שבטור: MS L שהוא בטור</ref> השפל | ||
|- | |- | ||
| − | | | + | |After you have done this and less remains in the upper row than in the bottom row and you want to check if your calculation was performed without making any mistakes, add up all the multiplication rows you received, each with its type and also what remains in the upper row and perform the addition operation, the procedure of which you are familiar with. If the sum is equal to the number you first had in the upper row, the calculation is correct, and if you find any change between them, there is an error. |
| − | + | |style="text-align:right;"|וכאשר עשית זה ונשאר בטור העליון פחות ממה שבטור השפל ותרצה לבחון אם נעשית מלאכתך בזולת נפילת בה שום טעות תחבר כל טורי הכפל אשר יצאו לך כל מין עם מינו וגם הנשאר לך בטור עליון ותנהוג מנהג החבור אשר ידעת מנהגו ואם המחובר יהיה שוה למספר שיהיה לך ראשונה בטור העליון הנה החשבון אמיתי ואם תמצא שום התחלפות ביניהם הנה טעות ודוק ותשכח‫<ref>עשית: MS L עשיתה<br> | |
| − | + | העליון: MS P1081 עליון<br> | |
| − | |- | + | שבטור: MS L שנשמר בטור<br> |
| − | | | + | נעשית מלאכתך: MS L מלאכתך נעשית<br> |
| + | בזולת: MS L מבלי<br> | ||
| + | בה: MS L om.<br> | ||
| + | תחבר: MS P1081 תסדר<br> | ||
| + | יצאו: Guenzburg שאו<br> | ||
| + | כל: MS L om.<br> | ||
| + | וגם: MS L גם<br> | ||
| + | מנהג: MS L om.<br> | ||
| + | ידעת מנהגו: MS L om.<br> | ||
| + | העליון: MS P1081 עליון<br> | ||
| + | הנה: MS L om.<br> | ||
| + | החשבון: MS L חשבונך<br> | ||
| + | שום התחלפות: MS L om.<br> | ||
| + | הנה: MS L om.</ref> | ||
| + | |- | ||
| + | |Table in MS Firenze <math>\scriptstyle{\color{red}{8}}894321\div94932</math> | ||
| + | | | ||
|- | |- | ||
| − | | | + | |colspan="2" | |
| − | + | {|class="wikitable" style="color:blue; text-align:center; | |
|- | |- | ||
| + | |the remainder of division | ||
| | | | ||
{|style="margin-left: auto; margin-right: 0px;" | {|style="margin-left: auto; margin-right: 0px;" | ||
|- | |- | ||
| − | | | + | |0||6||5||6||4||5 |
| + | |} | ||
|- | |- | ||
| − | | | + | |the second dividend |
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
|- | |- | ||
| − | | | + | |3||5||0||4||4||1 |
|} | |} | ||
|- | |- | ||
| + | |the result of division | ||
| | | | ||
{|style="margin-left: auto; margin-right: 0px;" | {|style="margin-left: auto; margin-right: 0px;" | ||
|- | |- | ||
| − | | | + | |8||9||4||3||2||1 |
| + | |- | ||
| + | | || || || ||9||3 | ||
| + | |- | ||
| + | | ||9||4||9||3||2 | ||
|} | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
| + | |- | ||
| + | |the upper row, which is the first dividend | ||
| + | |- | ||
| + | |the middle row, which is the quotient | ||
|- | |- | ||
| + | |the bottom row, which is the divisor | ||
|} | |} | ||
|- | |- | ||
| | | | ||
| − | + | {|style="margin-left: auto; margin-right: 0px;" | |
| − | |style=" | ||
|- | |- | ||
| − | | | + | |The product of the first digit of the quotient by the divisor |
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |The product of the second digit of the quotient by the divisor |
| − | |||
| − | |||
|- | |- | ||
| + | |The remainder of division to be added to the multiplication rows for the scales | ||
| + | |} | ||
| | | | ||
| − | + | {|style="margin-left: auto; margin-right: 0px;" | |
| − | |style=" | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |8||5||4||3||8||8|| |
| − | |||
| − | | | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |0||2||8||4||7||9||6 |
| − | |||
| − | | | ||
| − | |||
|- | |- | ||
| − | | | + | |0||0||6||5||6||4||5 |
| − | + | |} | |
| − | | | ||
| − | |||
|- | |- | ||
| + | |After the adding the bottom row, which is the remainder of the division above, the result is the sum of the three rows, and if the division is correct it is the original dividend according to the scales. | ||
| | | | ||
| − | + | {|style="margin-left: auto; margin-right: 0px;" | |
| − | |style=" | ||
|- | |- | ||
| − | | | + | |8||9||4||3||2||1 |
| − | + | |} | |
| − | | | ||
|- | |- | ||
| − | | | + | |} |
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |colspan="2" | |
| − | + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | |
| − | |style="text-align: | ||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| + | |הנשאר לחלק | ||
| | | | ||
| − | + | {|style="margin-left: auto; margin-right: 0px;" | |
| − | |style=" | ||
|- | |- | ||
| − | | | + | |0||ו||ה||ו||ד||ה |
| − | | | + | |} |
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| + | |המחולק השני | ||
| | | | ||
| − | |style=" | + | {|style="margin-left: auto; margin-right: 0px;" |
|- | |- | ||
| − | | | + | |ג||ה||0||ד||ד||א |
| − | | | + | |} |
|- | |- | ||
| + | |מגיע לכל חלק לפי ערך החילוק | ||
| | | | ||
| − | + | {|style="margin-left: auto; margin-right: 0px;" | |
| − | |style=" | ||
|- | |- | ||
| − | | | + | |ח||ט||ד||ג||ב||א |
| − | |||
| − | | | ||
|- | |- | ||
| − | | | + | | || || || ||ט||ג |
| − | |||
|- | |- | ||
| + | | ||ט||ד||ט||ג||ב | ||
| + | |} | ||
| | | | ||
{|style="margin-left: auto; margin-right: 0px;" | {|style="margin-left: auto; margin-right: 0px;" | ||
|- | |- | ||
| − | | | + | |הטור העליון ורק הוא המחולק ראשון |
|- | |- | ||
| − | | | + | |הטור האמצעי והוא החילוק |
|- | |- | ||
| − | | | + | |הטור השפל והוא המחלק |
| − | |||
| − | |||
| − | |||
|} | |} | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
{|style="margin-left: auto; margin-right: 0px;" | {|style="margin-left: auto; margin-right: 0px;" | ||
|- | |- | ||
| − | | | + | |הכפל מאות ראשונה מן החלוק עם המחלק |
|- | |- | ||
| − | | | + | |הכפל מאות שנייה מן החלוק עם המחלק |
|- | |- | ||
| − | | | + | |הנשאר לחלק כדי לצרפו עם כל טורי הכפל לעשות המאזניים |
| − | |||
| − | |||
|} | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: 0px;" | ||
|- | |- | ||
| − | | | + | |ח||ה||ד||ג||ח||ח|| |
|- | |- | ||
| − | | | + | |0||ב||ח||ד||ז||ט||ו |
| − | | | ||
|- | |- | ||
| − | | | + | |0||0||ו||ה||ו||ד||ה |
| − | | | + | |} |
|- | |- | ||
| + | |אחרי הצירוף מהטור הזה תחתון והוא הנשאר לחלק לעיל יעלה מחובר מהג' טורין אם החלוק אמתי וצודק והם למאזניים כשצודק עם המחולק בראשונה אם לא | ||
| | | | ||
| − | |style=" | + | {|style="margin-left: auto; margin-right: 0px;" |
|- | |- | ||
| − | | | + | |ח||ט||ד||ג||ב||א |
| − | | | + | |} |
|- | |- | ||
| − | | | + | |} |
| − | |||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |MS Firenze margin: |
| − | |style="text-align:right;"| | + | :An explanatory supplement that does not belong to this section: |
| + | |style="text-align:right;"|שלא מן המאמ' והוא תוס' ביאור | ||
|- | |- | ||
| − | | | + | |If you want to further divide the remainder from division in the procedure we did, decompose the remainder into the preceding rank. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואם תרצה לחלק עוד הנשאר לחלק בזה הדרך שעשינו שבר הנשאר למעלה ולמדרגה שלפניה |
|- | |- | ||
| − | | | + | |I.e. if the remainder are minutes, decompose them into seconds, or into thirds if the former were seconds, or to fourths, if they were thirds, and so on as long as the calculation continues. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ר"ל אם הנשאר הם מותר מדקים שברם לשניים וכן לשלישיים אם הקודמי' היו שניים וכן לרביעיים אם קדמו השלישיים וכן עד כולם כל אשר יימשך החשבון |
|- | |- | ||
| − | | | + | |Look at the previous division table and you will find the explanation of what the results are. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ועיין בלוח הקודם מהחילוק ותמצא ביאור העולים מה יהיו |
|- | |- | ||
| | | | ||
| − | + | ||
| − | + | == Extracting roots == | |
| | | | ||
| − | |||
|- | |- | ||
| − | | | + | |After I have explained to you the method of division in the easiest possible way, in addition to mentioning most of the number operations such as division, multiplication and subtraction, as well as addition and checking, I intend to clarify and inform an easy way to find the square roots of the numbers that have a real root, or the closest to the numbers that do not have a real root, whether they are integers alone, integers with fractions, or fractions alone; their rules and approximations, while mentioning the other methods of calculation also. |
| − | |style="text-align:right;"| | + | |style="width:45%; text-align:right;"|‫<ref>L: אמר החכם הנז‫'</ref><big>אחר</big> שביארתי לך דרך החלוק על הדרך היותר קל שאיפשר מצורף מה שיש בו מן ההזכרה מרוב דרכי המספר כמו חלוק כפל ומגרעת גם החיבור בבחינה אמרתי לבאר ולהודיע דרך קל למצא שרשי המרובעים מהמספרים שיש להם שורש אמיתי או היותר קרוב להם מהמספרים אשר אין להם שורש אמיתי שלמי' לבדם הן מהמספרים שיש שלמים עם נשברים או מנשברים לבדם הן וכלליהם ודקדוקהם ויהיה גם זה מן ההזכרה בשאר דרכי החשבון‫<ref>לך: L om.<br> |
| − | + | מה שיש בו: L om.<br> | |
| − | + | מן ההזכרה: L מההזכרה<br> | |
| − | + | היותר: P1081 margin<br> | |
| + | להם: MS L om.<br> | ||
| + | אשר אין: MS L שאין<br> | ||
| + | שלמים: MS L שברים<br> | ||
| + | נשברים: MS L השברים<br> | ||
| + | או: MS L הן<br> | ||
| + | לבדם: MS L om.<br> | ||
| + | מן ההזכרה: MS L מהזכרה</ref> | ||
|- | |- | ||
| − | | | + | |From now on I will start and say: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ומעתה אתחיל ואומר‫<ref>ומעתה: MS L ומזה</ref> |
|- | |- | ||
| − | | | + | |Know that in the rank of units there are three square numbers, i.e. whose roots are known integers and they are: one, whose root is one; four, whose root is two; and nine, whose root is three. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{\sqrt{1}=1\quad\sqrt{4}=2\quad\sqrt{9}=3}}</math> |
| + | |style="text-align:right;"|<big>דע</big> כי במעלת האחדים יש בה שלשה מספרים מרובעים ר"ל ששרשיהם שלמים וידועים והם אחד ששרשו אחד וארבעה ששרשו שנים ותשעה ששרשו שלשה‫<ref>במעלת: MS L במעלות<br> | ||
| + | בה: MS P1081 בו<br> | ||
| + | וארבעה: MS L ארבעה</ref> | ||
|- | |- | ||
| − | | | + | |The second rank, which is the rank of tens, does not have a square number at all, only together with units, because ten is not a square number, nor twenty, nor 30, nor 40, nor 50, 60, 70, 80, 90. Yet with the addition of units, it has six squares, i.e. we add to the number of tens some units: like 16, whose root is 4; 25, whose root is 5; 36, whose root is 6; 49, whose root is 7; 64 whose root is 8; 81, whose root is 9. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ובמעלה השנית שהיא מעלת עשרות אין בה מספר מרובע כלל רק עם חבור אחדים כי עשרה אינו מספר מרובע ולא עשרים ולא ל' ולא מ' ולא נ' ס' ע' פ' צ' אמנם עם חבור אחדים יש בה ששה מרובעים ר"ל שנוסיף על מספר העשרות אחדים מה כמו י"ו שרשו ד' כ"ה שרשו ה' ל"ו שרשו ו' מ"ט שרשו ז' ס"ד שרשו ח' פ"א שרשו ט‫'‫<ref>ובמעלה: MS P1081 והמעלה<br> |
| − | + | השנית: MS L שינית<br> | |
| − | + | שהיא מעלת עשרות: MS L om.<br> | |
| − | + | בה: MS L בו<br> | |
| − | |- | + | כלל: MS L om.<br> |
| − | | | + | עם: MS L om.<br> |
| − | + | ולא: MS L לא<br> | |
| + | MS L: אין בהם שום מספר מרובע<br> | ||
| + | אמנם: MS L רק<br> | ||
| + | אחדים: MS L האחדים<br> | ||
| + | בה: MS L בו<br> | ||
| + | ר"ל: Guenzburg ול"י<br> | ||
| + | שנוסיף על מספר: MS L שנשיב אחור<br> | ||
| + | אחדים מה: MS L ונחברם עם אחדים<br> | ||
| + | שרשו: MS L ששורשו five times</ref> | ||
| + | |- | ||
| + | |colspan="2" | | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{16}=4\quad\sqrt{25}=5\quad\sqrt{36}=6\quad\sqrt{49}=7\quad\sqrt{64}=8\quad\sqrt{81}=9}}</math> | ||
|- | |- | ||
| − | | | + | |Every rank of a number, whose numerical position is odd, such as third, fifth, seventh, ninth, and the like endlessly, is analogous to the rank of units. |
| − | |style="text-align:right;"|וכן | + | |style="text-align:right;"|וכל מעלת המספר שמספר מדרגתה נפרד כמו שלישית חמישית שביעית תשיעית ודומיהן לאין תכלית דומה למעלה האחדים‫<ref>מעלת: P1081: מעלות; L: מעלה ממעלות<br> |
| + | ודומיהן: MS L וכן<br> | ||
| + | למעלה: MS L למעלו‫'</ref> | ||
|- | |- | ||
| − | | | + | |As, if you say, for example, the rank of hundreds, which is the third rank, also has three squares: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כאלו תאמר דרך משל במעלת המאיות שהיא מדרגת שלישית יש בה גם כן שלשה מרובעים‫<ref>במעלת: MS L במעלות<br> |
| + | המאיות: MS L <s>האחדי'</s> המאיות<br> | ||
| + | מדרגת: MS L om.<br> | ||
| + | גם כן: MS L om.</ref> | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :A hundred, which is analogous to one, whose root is ten, which is analogous to one. |
| + | :<math>\scriptstyle{\color{blue}{\sqrt{100}=10}}</math> | ||
| + | |style="text-align:right;"|והוא מאה שדומה לאחד שרשו עשרה שדומה לאחד‫<ref>שדומה: Guenzburg ששרשו</ref> | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Four hundreds, which is analogous to four units, whose root is twenty, which is analogous to two. |
| + | :<math>\scriptstyle{\color{blue}{\sqrt{400}=20\quad\sqrt{4}=2}}</math> | ||
| + | |style="text-align:right;"|וארבע מאות שדומה לארבע אחדים שרשו עשרים שדומה לשנים‫<ref>וארבע: MS L ד‫'<br> | ||
| + | אחדים: MS L om.</ref> | ||
|- | |- | ||
| | | | ||
| − | + | :9 hundreds, which is analogous to 9 units, whose root is thirty, which is analogous to three. | |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{\sqrt{900}=30\quad\sqrt{9}=2}}</math> |
| + | |style="text-align:right;"|וט' מאות שדומה לט' אחדים שרשו שלשים שדומה לשלש‫<ref>וט‫': MS L ט‫'<br> | ||
| + | אחדים: MS L om.<br> | ||
| + | לשלש: Guenzburg לשלש הם; MS L: וכן כל מעלה שמספר מדרגתה נפרד כמו חמישי' ושביעי' ותשיעי' וכן לאין קץ</ref> | ||
| + | |- | ||
| + | |Likewise every rank whose numerical position is even, like fourth, sixth, eighth, tenth, and so on endlessly, which is called an even rank, is analogous to the rank of tens, which has six squares by adding units to it as we have explained. So, the rank of thousands has six squares by adding hundreds, i.e. we shift each thousand backward as ten hundreds and add them to what is in the hundreds: | ||
| + | |style="text-align:right;"|וכן כל מעלה שמספר מדרגתה זוג כמו רביעית ששית שמינית עשירית וכן לאין תכלית והיא אשר תקרא מעלה זוגיית דומה למעלה העשרות שיש בה ששה מרובעים עם חבור אחדים בה כמו שבארנו כן במעלת האלפים יש בה ששה מרובעים עם חבור המאיות ר"ל שנשים כל אלף ואלף אחורנית לעשרה מאיות ותחבר עם מה שנמצא במאיות‫<ref>וכן כל: MS L וכל<br> | ||
| + | שמספר: MS L om.<br> | ||
| + | והיא: MS P1081 והוא<br> | ||
| + | מעלה: MS L מעלת<br> | ||
| + | זוגיית: MS L זוגות<br> | ||
| + | בה מרובעים ... יש בה: MS L om.<br> | ||
| + | המאיות: MS L <s>עשרות</s> מאיות<br> | ||
| + | שנשים: MS L שנשיב<br> | ||
| + | ואלף: MS L אלף<br> | ||
| + | ותחבר: MS L ונסדר<br> | ||
| + | עם: MS L כל</ref> | ||
|- | |- | ||
| | | | ||
| − | ::{| | + | :Like one thousand and six hundred, which is analogous to sixteen, whose root is forty, which is analogous to four. |
| + | :<math>\scriptstyle{\color{blue}{\sqrt{1600}=40\quad\sqrt{16}=4}}</math> | ||
| + | |style="text-align:right;"|כמו אלף ושש מאות הדומה לשש <ref>Guenzburg 194v</ref>עשרה שרשו ארבעים הדומה לארבעה‫<ref>עשרה: MS L עשר<br> | ||
| + | לארבעה: MS L לארבע</ref> | ||
|- | |- | ||
| | | | ||
| − | {|style=" | + | :Two thousands and 500, which is analogous to 25, whose root is fifty, which is analogous to five. |
| + | :<math>\scriptstyle{\color{blue}{\sqrt{2500}=50\quad\sqrt{25}=5}}</math> | ||
| + | |style="text-align:right;"|ואלפים ות"ק הדומה לכ"ה שרשו חמשים הדומה לחמשה | ||
| + | |- | ||
| + | | | ||
| + | :Three thousand and six hundred, which is analogous to 36, whose root is sixty, which is analogous to six. | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{3600}=60\quad\sqrt{36}=6}}</math> | ||
| + | |style="text-align:right;"|ושלשת אלפים ושש מאות הדומה אל ל"ו שרשו ששים הדומה לששה‫<ref>לל"ו: MS P1081 ל"ו<br> | ||
| + | ששים הדומה: MS L om.<br> | ||
| + | לששה: MS L ו‫'</ref> | ||
|- | |- | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| | | | ||
| − | {| | + | :Four thousand and nine hundred, which is analogous to 49, whose root is seventy, which is analogous to seven. |
| + | :<math>\scriptstyle{\color{blue}{\sqrt{4900}=70\quad\sqrt{49}=7}}</math> | ||
| + | |style="text-align:right;"|וארבעה אלפים ותשע מאות שדומה למ"ט שרשו שבעים שדומה לשבעה | ||
|- | |- | ||
| | | | ||
| − | {|style=" | + | :Six thousand and four hundred, which is analogous to 64, whose root is eighty, which is analogous to eight. |
| + | :<math>\scriptstyle{\color{blue}{\sqrt{6400}=80\quad\sqrt{64}=8}}</math> | ||
| + | |style="text-align:right;"|וששת אלפים ארבע מאות הדומה לס"ד שרשו שמנים הדומה לשמנה | ||
|- | |- | ||
| − | | | + | | |
| + | :Eight thousand and one hundred, which is analogous to 81, whose root is ninety, which is analogous to nine. | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{8100}=90\quad\sqrt{81}=9}}</math> | ||
| + | |style="text-align:right;"|ושמנה אלפים ומאה הדומה לפ"א שרשו תשעים הדומה לתשעה‫<ref>וח' אלפים ומאה: MS P1081 ומאה אלפים ושמנה</ref> | ||
|- | |- | ||
| − | | | + | |And so on every rank whose numerical position is even, like fourth, sixth, eighth, to infinity. |
| − | + | |style="text-align:right;"|וכן כל מעלה שמספר מדרגתה זוג כמו רביעית ששית שמינית לאין תכלית‫<ref>וכן: Guenzburg וכל<br> | |
| + | שמינית: Guenzburg שמנים</ref> | ||
|- | |- | ||
| − | | | + | |You should know that the result of any number falls in the middle rank of the ranks of that number. |
| + | |style="text-align:right;"|ולך לדעת כי היוצא מכלל איזה מספר שיהיה יפול במעלת האמצעית שבמספר המדרגות‫<ref>במעלת: Guenzburg במעלת במעלת; MS P1081 המעלה<br> | ||
| + | האמצעית: MS P1081 הממוצעת</ref> | ||
|- | |- | ||
| | | | ||
| − | : | + | *As, if you say, for example: we have the number 4 in the seventh rank, which is an odd rank, like in this diagram: |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{\sqrt{4000000}}}</math> |
| − | + | |style="text-align:right;"|כאלו תאמר דרך משל יש לנו מספר ד' במעלה השביעית שהוא מעלה נפרדת כמו הענין בזאת הצורה‫<ref>מספר: MS L om.<br> | |
| − | + | הענין: MS L om.</ref> | |
| − | : | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| Line 1,117: | Line 1,115: | ||
{|style="margin-left: auto; margin-right: 0px;" | {|style="margin-left: auto; margin-right: 0px;" | ||
|- | |- | ||
| − | | | + | |4||0||0||0||0||0||0 |
|- | |- | ||
| − | | || || || | + | | || || ||2|| || || |
|} | |} | ||
|} | |} | ||
| Line 1,128: | Line 1,126: | ||
{|style="margin-left: auto; margin-right: 0px;" | {|style="margin-left: auto; margin-right: 0px;" | ||
|- | |- | ||
| − | | | + | |ד||0||0||0||0||0||0 |
|- | |- | ||
| − | | || || || | + | | || || ||ב|| || || |
|} | |} | ||
|- | |- | ||
| Line 1,136: | Line 1,134: | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Its root is 2. As we explained, 2 should be written in the fourth rank, which is the middle rank that has 3 rank before it and the same after it. |
| + | |style="text-align:right;"|ושרשו ב' כמו שביארנו שראוי לכתוב ב' במעלה הרביעית שהיא מעלה האמצעית שיש לפניה ג' מדרגות וכהנה לאחריה‫<ref>ושרשו: MS L שרשו<br> | ||
| + | ב‫': Guenzburg om.<br> | ||
| + | הרביעית: MS L רביעית כמו העניין בצורה; Guenzburg om.<br> | ||
| + | המעלה: MS L למעלה</ref> | ||
|- | |- | ||
| − | | | + | |Understand it for the other odd ranks. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ובין תבין בשאר המעלות הנפרדות |
|- | |- | ||
| − | | | + | |The same for the even rank: as we explained, there is no square in it, unless we return the last digit in the even rank backward, so each unit becomes a ten and we add everything to what is in the preceding rank. Then, the root should be extracted. The number of the ranks becomes odd, and every odd rank has a middle rank. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וגם במעלה הזוגיית כמו שבארנו אין בה שום מרובע אם לא בהשיב כל אחד מהמספר האחרון שבמעלה הזוגיית אחורנית ולעשות מכל אחד עשרה ולחבר הכל עם מה שבמעלה שלפניה ואז ראוי לקחת השרש ואז ישובו מספרי המעלות נפרד וכל נפרד יש לה מעלה אמצעית‫<ref>כמו שבארנו: MS P1081 om.<br> |
| + | אחד: MS P1081 om.<br> | ||
| + | שבמעלה: Guenzburg <s>שמ</s> שבמעלה<br> | ||
| + | ואז: MS P1081 ואם<br> | ||
| + | ישובו: MS P1081 יש בו</ref> | ||
|- | |- | ||
| − | | | + | |The resulting root should be written in the middle rank, i.e. as far from the last rank, from which it is extracted, as its distant from the units, because the units stand by themselves, in their rank and the root of the units is in the rank of the units. |
| − | ::{|class="wikitable" style="color:blue; text-align:center;" | + | |style="text-align:right;"|וראוי לכתוב השרש היוצא במדרגת האמצעית ר"ל רחוק ממדרגה האחרונה אשר ילקח מהם כאשר מרחקו מן האחדים מפני שהאחדים עומדים בעצמם ובמקומם והוא שרש האחדים בטור האחדים‫<ref>האחרונה: MS L <s>הראשונה</s> האחרונה<br> |
| + | אשר ילקח: MS L שילקח<br> | ||
| + | בטור: MS P1081 ביאור</ref> | ||
| + | |- | ||
| + | |The meaning is that the root is as far from the rank from which it is extracted as it is far from the units, i.e. the distance from the units is the same. | ||
| + | |style="text-align:right;"|והענין אחר שהשרש <s>נקו</s> רחוק ממדרגת אשר לוקח ממנו כאשר ירחק מן האחדים ר"ל שזה וזה לא ירחקו דבר מן האחדים‫<ref>אשר לוקח: MS L שלוקח<br> | ||
| + | ר"ל שזה וזה: MS L נ‫'<br> | ||
| + | מן האחדים: MS L מהאחדים</ref> | ||
| + | |- | ||
| + | |After I have introduced this to you, I remind you the arrangement of the squares that are in the rank of units, which is the first: 1, 4, 9, whose roots are: 1, 2, 3 and in the rank of tens there are six squares: 16, 25, 36, 49, 64, 81 and their roots are: 4, 5, 6, 7, 8, 9. This is already known to you. | ||
| + | |style="text-align:right;"|ואחר שהקדמתי לך זה אזהירך להזכירך מסורת המרובעים אשר במדרגת האחדים שהיא הראשונה שהם א' ד' ט' ושרשם א' ב' ג' ובמדרגות העשרות יש ששה מרובעים והם י"ו כ"ה ל"ו מ"ט ס"ד פ"א ושרשם ד' ה' ו' ז' ח' ט' ויהיה זה ידוע אצלך‫<ref>זה: MS L om.<br> | ||
| + | אזהירך: MS L אזכירך<br> | ||
| + | להזכירך: MS L להזהירך<br> | ||
| + | במדרגת: MS P1081 במדרגות<br> | ||
| + | א‫': MS L ב‫'<br> | ||
| + | ד‫': MS P1081 א‫'<br> | ||
| + | פ"א: MS P1081 פ"א פ"א</ref> | ||
| + | |- | ||
| + | |I have already informed you that the odd rank follows the rule of the units and the even [rank] follows the rule of the tens. | ||
| + | |style="text-align:right;"|וכבר הודעתיך כי המעלה הנפרדת הנה דינה כדין האחדים והזוגית דינה כדין העשרות‫<ref>כי המעלה הנפרדת הנה: MS L om.</ref> | ||
| + | |- | ||
| + | |Now I will teach you the procedure you apply in order to find the root of any number you want, whether it has a real root or the closest to it, if it does not have a real root: | ||
| + | |style="text-align:right;"|ועתה אורה אותך דרך זו תלך בידיעת שרש איזה מספר שתרצה אם יש להם שרש [אמתי] או היותר קרוב לפניו אם אין להם שרש אמיתי‫<ref>בידיעת: MS P1081 וידיעת<br> | ||
| + | שרש: MS P1081 השורש<br> | ||
| + | להם: MS L לו</ref> | ||
| + | |- | ||
| + | |Write the number, whose root you want to know, in one row by its ranks as many as they may be. | ||
| + | |style="text-align:right;"|הנה תכתוב המספר אשר תרצה לדעת שרשו בטור אחד איזו מדרגות שתהיינה‫<ref>אשר תרצה: MS L שתרצה</ref> | ||
| + | |- | ||
| + | | | ||
| + | *Suppose first that it has five ranks, for example: 12345. | ||
| + | |style="text-align:right;"|ותאמר תחלה שתהיינה ה' מדרגות דרך משל א'ב'ג'ד'ה'‫<ref>א' ... ה‫': MS L om.</ref> | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color:blue; text-align:center;" | ||
|- | |- | ||
| | | | ||
| Line 1,171: | Line 1,211: | ||
|- | |- | ||
| ||1||3||8||9 | | ||1||3||8||9 | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|} | |} | ||
|- | |- | ||
| Line 1,215: | Line 1,247: | ||
|- | |- | ||
| ||א||ג||ח||ט | | ||א||ג||ח||ט | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|} | |} | ||
|- | |- | ||
| Line 1,234: | Line 1,258: | ||
|- | |- | ||
| | | | ||
| − | : | + | ::The last rank is odd, so it follows the rule of the units. Therefore, we consider the last digit 5 as if it is 5 units. The closest preceding root is 2, which is the root of 4. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\sqrt{4}=2}}</math> |
| + | |style="text-align:right;"|הנה המעלה האחרונה היא נפרדת ודינה כדין האחדים לכן נדמה מספר ה' האחרון כאלו הוא ה' אחדים הנה שרשו היותר קרוב לו לפניו הוא ב' שהוא שרש ד‫'‫<ref>ה‫': MS L om.<br> | ||
| + | הוא: MS L om.<br> | ||
| + | ה‫': MS P1081 om.<br> | ||
| + | לו: MS L om.<br> | ||
| + | שורש: MS L om.</ref> | ||
|- | |- | ||
| | | | ||
| − | : | + | ::Therefore, we write 2 in the middle, beneath the digit 3, and we draw a line beneath the 2 with some space, so that we can multiply the resulting digit of the root. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|לכן נכתוב ב' באמצע שהוא תחת אות הג' ונרשום קו תחת הב' עם מעט ריוח כדי שנוכל לכפול אות השרש היוצאת‫<ref>הג‫': MS L ג‫'<br> |
| + | ונרשום: MS L וירשום<br> | ||
| + | ריוח: MS L om.<br> | ||
| + | אות השרש: MS L האותיות</ref> | ||
|- | |- | ||
| | | | ||
| − | : | + | ::We multiply the digit 2, which is the root, by itself. The product should be written following it, as far from it as it is far from the units. Zeros should be written as the number of the ranks before it, starting straightly corresponding to the 2 in its rank, as we say now corresponding to the 2, we start writing 2 zeros, as the digit 2 is far from the units by two ranks. After the 2 zeros, we multiply the 2 by itself. Understand and remember it for every product. Then, subtract the product from the first corresponding digit, as known in the subtraction procedure, and the remainder remains above the upper row. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{OliveGreen}{5-2^2=5-4=1}}</math> |
| + | |style="text-align:right;"|ונכפול אות הב' שהוא השרש בעצמו והנה ראו[י] לכתוב הכפולה רחוק ממנה אחריה כאשר היא רחוקה מן האחדים וראוי לכתוב גלגלים כמספר המדרגות אשר לפניה והתחלתם כנגד הב' ביושר ובמדרגתה כאשר נאמר עתה כנגד הב' נתחיל ‫<ref>195r</ref>לכתוב ב' גלגלים כאשר אות הב' רחוקה מן האחדים שני מדרגות ואחר השני גלגלים נכפול הב' בעצמה ויהיה ד' ובין תבין בכל התחלת הכפולה וזכור לך זה ואחר גרע העולה מהכפל מהמספר הראשון מן במינו כאשר נודע המגרעת והנשאר ישאר על הטור העליון‫<ref>ממנה: MS L om.<br> | ||
| + | ולכתוב ... מן האחדים: MS L om.<br> | ||
| + | שני: MS P1081 שבו<br> | ||
| + | מדרגות: MS L המדרגות<br> | ||
| + | התחלת: MS L התחלה<br> | ||
| + | מהמספר: MS L המספר<br> | ||
| + | במינו: MS P10181 ממינו<br> | ||
| + | ישאר: MS L יהיה</ref> | ||
|- | |- | ||
| | | | ||
| − | : | + | ::Then, multiply the root by two, i.e.: if it is 3, write 6 beneath it; if it is 4, [write] 8. In this example, it is 2, so write 4 and this is the root multiplied by two. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{2\times2=4}}</math> |
| + | |style="text-align:right;"|אחר תכפול השרש בשנים ר"ל שאם היה ג' תכתוב תחתיה ו' ואם ד' ח' ובדמיונו זה שהיה ב' תכתוב ד' והוא השרש הכפול בשנים‫<ref>ג‫': MS P10181 om.<br> | ||
| + | תכתוב: MS L תכפול<br> | ||
| + | תחתיה: MS L תחתיו<br> | ||
| + | ואם: MS L <s>והאם</s> ואם<br> | ||
| + | שהיה: MS L שאם היה<br> | ||
| + | השרש הכפול: MS L שורש הכפל<br> | ||
| + | בשנים: MS L om.</ref> | ||
|- | |- | ||
| | | | ||
| − | : | + | ::Divide the remainder of the first digit by this 4: take as much as you can, so that you can take the square of the quotient. As you say in this example: we return the 1 back, because we cannot take 4 from it even once. We return it back to the 4 that precedes it; it is 14. We say: how many times can we take 4 from 14? We take 3, because we can take 3 times 3, which is the square of the quotient, from what is left before. We write 3 before the first root. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|תחלק הנשאר מהמספר הראשון על זאת הד' ותקח כל מה שתוכל באופן שתוכל לקחת מרובע המספר אשר יצא מהחלוקה כאלו תאמר בדמיונו זה הנה נשוב הא' אחורנית כי לא נוכל לקחת ד' ממנו אפי' פעם אחד ונשיבהו אחורנית עם הד' אשר לפניה ויהיה י"ד ונאמר כמה פעמים נוכל לקחת ד' מן י"ד הנה נקח ג' כי מהנשאר לפניו נוכל לקחת ג' פעמים ג' שהוא מרובע המספר היוצא מחלוקה ונכתוב ג' לפני השרש הראשון‫<ref>מהמספר הראשון: MS L om.<br> |
| + | זאת: MS L אות<br> | ||
| + | תאמר: MS P1081 אומר<br> | ||
| + | ונשיבהו: MS L והשיבו ה <s>עם הד‫'</s><br> | ||
| + | נוכל: MS L תוכל<br> | ||
| + | לקחת: Guenzburg om.<br> | ||
| + | המספר: MS L om.</ref> | ||
|- | |- | ||
| | | | ||
| − | : | + | ::We multiply the 3 first by itself and then by the doubled root. |
| − | + | |style="text-align:right;"|ונכפול זאת הג על עצמה ראשונה ואחר על השרש הכפול‫<ref>זאת הג‫': MS L ג‫'<br> | |
| − | |style="text-align:right;"| | + | ואחר על: MS L ועל</ref> |
|- | |- | ||
| | | | ||
| − | : | + | ::We start with the 1 next to it: write as many zeros as the distance of the digit 3 from the units; it is one zero corresponding to the 3. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ונתחיל לכתוב זאת האות הנופלת א' בצדה ותעשה ראשונה גלגלים כאשר אות הג' רחוקה מהאחדים והוא גלגל אחד ראשונה כנגד הג‫'<ref>ונתחיל: MS L ותתחיל<br> |
| + | הנופלת: MS P1081 הכופלת</ref> | ||
|- | |- | ||
| | | | ||
| − | : | + | ::We say: 3 times 3 is 9. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{3\times3=9}}</math> |
| + | |style="text-align:right;"|ואחר נאמר ג' פעמים ג' הם ט‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | ::We say: 3 times 4 is 12. Write 12. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{3\times4=12}}</math> |
| + | |style="text-align:right;"|ואחר נאמר ג' פעמים ד' הם ב'א' ותכתוב ב'א‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | ::We subtract the multiplied row from the the remainder above, each from the one that corresponds to it, according to the rule of subtraction, and what is left remains above in the upper row. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{OliveGreen}{143-129=14}}</math> |
| + | |style="text-align:right;"|ואחר נגרע זה הטור הנכפל מן הנשאר למעלה מן במינו כמשפט המגרעת והנשאר ישאר למעלה בטור העליון‫<ref>מן הנשאר: MS L מהנשאר<br> | ||
| + | במינו: MS L מן מינו<br> | ||
| + | העליון: MS P1081 עליון</ref> | ||
|- | |- | ||
| | | | ||
| − | : | + | ::Then, multiply the second digit of the root, which is 3, by two. We write 6 beneath it. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{2\times3=6}}</math> |
| + | |style="text-align:right;"|אחר זאת תכפול זאת האות השנית מן השרש שהיא ג' בשנים ונכתוב ו' תחתיה‫<ref>זאת: MS L om.<br> | ||
| + | זאת: MS P1081 זה<br> | ||
| + | השנית: MS P1081 השני<br> | ||
| + | מן השורש: MS L מהשורש<br> | ||
| + | שהיא: MS L שהוא</ref> | ||
|- | |- | ||
| | | | ||
| − | :We | + | ::We divide again all that remains above by the whole doubled root, which is 46. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{2\times23=46}}</math> |
| + | |style="text-align:right;"|<s>ונשב</s> ונשוב לחלקו כל הנשאר למעלה על כל השרש הנכפל שהוא ו'ד‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | ::We take as much as we can, so that we can take the square of the quotient from the last [digit]. As you say in this example: we return the 1 back, because we cannot take 4 from it; it is 14. We take 13, because we can take 3 times from the remainder and we can take 3 times 3 from the remainder after it. We write 3 before the 6, which is in the rank of the units. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ונקח כל מה שנוכל באופן שנוכל לקחת באחרונה מרובע המספר היוצא מן החלוקה כאלו תאמר בדמיונו זה הנה נשוב הא' אחורנית כי לא נוכל לקחת ד' ממנו ויהיו י"ד ונקח ג"א כי מהנשאר נוכל לקחת ג' פעמים ומהנשאר אחר זה נוכל לקחת ג' פעמים ג' ונכתוב ג' לפני הו' והיא במעלת האחדים‫<ref>באופן שנוכל: MS P1081 om.<br> |
| + | המספר: MS L om.<br> | ||
| + | מן החלוקה: MS L מהחלוקה<br> | ||
| + | והיא: MS P1081 והוא</ref> | ||
|- | |- | ||
| | | | ||
| − | : | + | ::First, we multiply this 3 by itself; it is 9. We write it corresponding to it, because the digit 3 is in the rank of the units. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{3\times3=9}}</math> |
| + | |style="text-align:right;"|ונכפול זאת הג' ראשונה בעצמה והוא ט' ונכתבנה כנגדה לפי שאות הג' היא במעלת האחדים‫<ref>ונכפול זאת הג' ... היא במעלת האחדים: MS P1081 om.</ref> | ||
|- | |- | ||
| | | | ||
| − | : | + | ::Next, we multiply 3 by 6 and 3 by 4. We subtract the products from what is left above and the remainder remains above. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אחר נכפול ג' על ו' ואחר ג' על ד' ואחר נגרע העולה מהכפל מן הנשאר למעלה והנשאר ישאר למעלה‫<ref>למעלה: MS L om.</ref> |
|- | |- | ||
| | | | ||
| − | : | + | ::The remainder above is 32 and the root is 233. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{OliveGreen}{1421-1389=32}}</math> |
| + | |style="text-align:right;"|וישאר למעלה ל"ב והשרש ג'ג'ב‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|‫ | + | :The explanation is complete. |
| + | |style="text-align:right;"|ונשלם באורו | ||
| + | |- | ||
| + | |I will give you various rules, the knowledge of which is necessary for the root extraction procedure. | ||
| + | |style="text-align:right;"|אך אמנם אתן לך כללים מתחלפים יש צורך בידיעתם במעשה השרשים‫<ref>אמנם: MS L om.</ref> | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *The first rule: when you extract the first root from the last digit you have, if it is in an odd rank, or in the preceding to the last, if it is in an even rank, you should write that root in the middle rank, as I have shown you and take as much as you can. If you cannot take anything from what remains in that last rank, and you take from the [rank] that is second to it, write it as far from the place, from which you take the quotient, as the first root is far from the units. So, you should write a zero in the place that precedes the first root, and before this zero you should write the result of the division. |
| + | |style="text-align:right;"|<big>הכלל הראשון</big> כאשר לקחת השרש הראשון מהמספר האחרון אשר בידך אם היא מדרגת נפרדת או מהקודם לאחרון אם הוא מדרגת זוגית ראוי לכתוב השרש ההוא במדרגת האמצעית כאשר הראיתיך ותקח כל מה שתוכל ואם לא תוכל לקחת דבר מהמקום ההוא האחרון הנשאר ולקחת מהשני לו גם כן תכתבהו רחוק מהמקום אשר תקח ממנו החלוק כאשר השרש הראשון רחוק מהאחדים ולכן ראוי לכתוב גלגל במקום אשר לפני השרש הראשון וקודם ‫<ref>195v</ref>לזה הגלגל תכתוב היוצא מן החלוקה‫<ref>האחרון: MS L הראשון<br> | ||
| + | גם כן: MS L ג"כ<br> | ||
| + | אשר תקח: MS L שתקח<br> | ||
| + | השרש: MS L ראש השורש<br> | ||
| + | אשר לפני: MS L שלפני</ref> | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *The second rule: when you divide again what remains of the number you have by double the root, do not take the last number from which you took it first, even if you see that it is possible to take from it, because when you take the root from it first, you already take from it everything that should [be taken from it], as you see in our example |
| + | |style="text-align:right;"|<big>הכלל השני</big> כאשר תשוב לחלק הנשאר מהמספר אשר בידך כפול השרש לא תקח המספר האחרון אשר לקחת ממנו ראשונה ואף כי מהמספר אשר אחריו ואף כי תראה כי האפשר לקחת בו מפני שכאשר לוקח ממנו השרש ראשונה כבר לוקח ממנו כל הראוי כמו שנראה בדמיונו זה‫<ref>תשוב: MS P1081 תשיב<br> | ||
| + | כפול: MS L כפלו<br> | ||
| + | אשר לקחת: MS L שלקחת<br> | ||
| + | ממנו: MS P1081 om.<br> | ||
| + | מהמספר: MS L ממספר<br> | ||
| + | אשר אחריו: MS L שאחריו<br> | ||
| + | תראה כי: MS L om.<br> | ||
| + | השורש: MS L השורש <s>הקרוב והוא</s> א<br> | ||
| + | כל: MS L om.<br> | ||
| + | כמו שנראה: MS L om.</ref> | ||
|- | |- | ||
| | | | ||
| − | : | + | ::{|class="wikitable" style="color:blue; text-align:center;" |
| − | |style="text-align: | ||
|- | |- | ||
| | | | ||
| − | + | {|style="margin-left: auto; margin-right: 0px;" | |
| − | |style=" | ||
|- | |- | ||
| − | | | + | |6|| || |
| − | |||
| − | | | ||
|- | |- | ||
| − | | | + | |3||9||9 |
| − | |||
| − | | | ||
|- | |- | ||
| − | | | + | | ||1|| |
| − | |||
| − | | | ||
|- | |- | ||
| + | | ||2|| | ||
| + | |} | ||
| + | |} | ||
| | | | ||
| − | + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | |
| − | |style="text-align: | ||
|- | |- | ||
| | | | ||
| − | + | {|style="margin-left: auto; margin-right: 0px;" | |
| − | |style=" | + | |- |
| + | |ו|| || | ||
| + | |- | ||
| + | |ג||ט||ט | ||
| + | |- | ||
| + | | ||א|| | ||
| + | |- | ||
| + | | ||ב|| | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
|- | |- | ||
| | | | ||
| − | + | *We wish to know the approximate root of 399. | |
| − | :<math>\scriptstyle{\color{blue}{ | + | :<math>\scriptstyle{\color{blue}{\sqrt{399}}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|רצינו לדעת השרש הקרוב אל ט'ט'ג‫'‫<ref>רצינו ... ט'ט'ג‫': MS L om.</ref> |
|- | |- | ||
| | | | ||
| − | : | + | ::We take the closest root, which is 1, and we write it in the middle rank, which is beneath the second 9. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והנה לקחנו השרש הקרוב והוא א' <s>וכתב</s> וכתבנוהו במדרגת האמצעית שהוא תחת הט' השניה |
|- | |- | ||
| | | | ||
| − | : | + | ::We subtract 1 from the last digit, which is 3; 2 remains above the 3. |
| − | :<math>\scriptstyle{\color{blue}{3 | + | ::<math>\scriptstyle{\color{blue}{3-1=2}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וגרענו א' מהמספר האחרון שהוא ג' ונשאר ב' על הג‫'‫<ref>רצינו ... לקחנו: Guenzburg twice<br> |
| + | מהמספר: MS L ממספר</ref> | ||
|- | |- | ||
| | | | ||
| − | : | + | ::We double the digit 1; it is 2. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{2\sdot1=2}}</math> |
| + | |style="text-align:right;"|ונכפול אות הא' והיא הב‫'‫<ref>והיא: MS L והוא</ref> | ||
|- | |- | ||
| | | | ||
| − | : | + | ::We do not say and look how many times the 2, which is double the root, is in the number that remained from the first number, which is 2, as we can take 2 from 2 once and then we can also take the square of 1 from the remainder. But this is not appropriate to do, because it would be appropriate to write the resulting 1 far from the number, from which we took the quotient, when the digit 1 written first is written according to this rule. Since we have already taken all that is needed from that rank, so we return the 2 back on the 9 that precedes it and it is 29. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ולא נאמר ולראות כמה פעמים יהיה ב' שהוא כפל השרש במספר הנשאר ממספר הראשון והוא ב' מאשר נוכל לקחת פעם אחת ב' מב' וגם נוכל לקחת מרובע א' מהנשאר זה אין ראוי לעשות מפני כי יהיה מהראוי לכתוב זה הא' היוצא רחוק מהמספר אשר לקחנו ממנו החלוק כאשר אות הא' הנכתב ראשונה נכתב כמשפט הזה וכבר לקחנו כל המצטרך במדריגה ההיא ולכן נשוב הב' אחורנית על הט' אשר לפניה ויהיה כ"ט‫<ref>הנשאר: MS L ב' הנשאר<br> |
| + | מהנשאר: MS P1081 מהראשון מהנשאר<br> | ||
| + | מפני כי: MS L ומפני זה<br> | ||
| + | יהיה: MS L om.<br> | ||
| + | אשר לקחנו: MS L שלקחנו<br> | ||
| + | הא‫': MS L הג‫'<br> | ||
| + | ראשונה: MS L ראשון<br> | ||
| + | נכתב: MS P1081 ב' נכתב<br> | ||
| + | כמשפט: MS L כמספר<br> | ||
| + | אשר לפניה: MS L שלפניה<br> | ||
| + | כ"ט: MS L om.</ref> | ||
|- | |- | ||
| | | | ||
| − | : | + | ::We say: how many times 2, which is double the root, is in the number 29, so we can take the square of the quotient from the preceding rank. |
| − | + | |style="text-align:right;"|ונאמר כמה פעמים יהיה ב' שהוא כפל השרש במספר כ"ט באופן שנוכל לקחת מהמדרגה שלפניה מרובע המספר היוצא מהחלוקה | |
| − | + | |- | |
| − | |style="text-align:right;"| | ||
| − | |- | ||
| | | | ||
| − | : | + | ::This is enough for this rule and it follows the third rule. |
| − | + | |style="text-align:right;"|ודי בזה זה הכלל והוא נמשך עם הכלל השלישי | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | : | + | *The third rule: when you divide again the remaining number by double the root, do not bother yourself to see if you can take ten times the divisor from the dividend, as stated in the example above: we see how many times 2 is in the number 29 and we say that it is ten, because this is impossible, even if it is more than ten, because you will not be able to take ten times ten from the preceding rank. It is not appropriate to bother with it, and this is also the rule of this matter. |
| − | + | |style="text-align:right;"|<big>הכלל השלישי</big> כאשר שבת לחלק המספר הנשאר על השרש הכפול לא תטריח בעצמך לראות אם תוכל לקחת עשרה פעמים המחלק במחולק כמו שנאמר בדמיונו שלמעלה מזה נראה כמה פעמים יהיה ב' במספר כ"ט ונאמר שיהיה בו עשרה כי זה אי אפשר ואף כי יותר מעשרה כי לא תוכל לקחת מהמדרגה שלפנינו עשרה פעמים עשרה ואין ראוי לטרוח בזה וכן זה הדין בענין החלוק‫<ref>המספר: MS L מספר<br> | |
| − | |style="text-align:right;"| | + | במחולק: MS P1081 במחלק<br> |
| + | יהיה: MS P1081 נקוה<br> | ||
| + | כי: MS L om.<br> | ||
| + | ואף כי יותר מעשרה: MS L om.<br> | ||
| + | זה: MS L om.</ref> | ||
|- | |- | ||
| | | | ||
| − | : | + | *The fourth rule: as we said in the second rule that when we divide the remainder again by double the root, we do not take again from the rank we took first, this is when we double the root and we do not add a rank to it, as if we double 2, it is 4 in the same rank, the same for 3 and 4. But, if the root is 5, or 6, or 7, or 8, or 9, when we double the root, one rank is added to the root. As if you say: the root is 6 tens, so double the root is 2 tens and one hundred, then one rank is added to the root. According to this, when you divide again, you take from the rank, from which you took first, and you write it also far from the last digit as the beginning of the doubled root is far from the units. This is what we wanted to clarify. |
| − | |style="text-align:right;"|וכן | + | |style="text-align:right;"|<big>הכלל הרביעי</big> מאשר אמרנו בכלל השני כי כאשר נשוב לחלק הנשאר על [כפל] השרש שלא נשוב לקחת מהמדרגה אשר לקחנו ראשונה זהו כאשר היה כשכפל[נו] זה השרש לא הוספנו עליו מדרגה כמו אם כפלנו ב' היה ד' באותה מדרגה וכן ג' וכן ד' אמנם אם היה השורש ה' או ו' או ז' או ח' או ט' והנה כשכפלנו זה השורש כבר נתוספה בשורש מדרגה אחת כאלו תאמר היה השורש ו' עשרות הנה כפל השורש ב' עשרות ומאה הנה ותוספת השרש מדרגת אחת ולפי זה כאשר תשוב לחלק תקח מהמדרגה אשר לקחת ממנו ראשונה ותכתבנה גם כן רחוקה מהמספר האחרון כאשר ראש השרש הכפול רחוק מן האחדים וזהו מה שרצינו לבאר‫<ref>נשוב: MS P1081 נשאר<br> |
| + | כשכפלנו: MS P1081 השפלנו<br> | ||
| + | הוספנו: MS L נוסף<br> | ||
| + | והנה: MS L הנה<br> | ||
| + | לא הוספנו עליו... כשכפלנו זה השורש: Guenzburg om.<br> | ||
| + | כבר: MS L om.<br> | ||
| + | נתוספה... ומאה הנה: Guenzburg om.<br> | ||
| + | ותוספת: MS P1081 נוספה<br> | ||
| + | לחלק: MS L om.<br> | ||
| + | גם כן: MS L ג"כ<br> | ||
| + | הכפול: MS L כפול<br> | ||
| + | מן האחדים: MS L מהאחדים</ref> | ||
|- | |- | ||
| | | | ||
| − | + | === Another Version - MS Paris 903 === | |
| − | + | ||
| − | |||
| | | | ||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |The way to extract a square root of a number that has a real root, or an approximate square root of those that do not have a real root, for integers alone, or for fractions alone, or for integers and fractions together. |
| − | + | |style="text-align:right;"|‫<ref>P903 138v</ref>הדרך להוציא שרשי המרובעי' ממספר בעל שרש אמתי או שרש המרוב' הקרוב מאשר אי' להן שרש אמתי | |
| − | |style="text-align:right;"| | + | הן משלמי' לבד או נשברי' לבד הן ממספר שלמי' ונשברי' יחד |
|- | |- | ||
| − | | | + | |Know that in the rank of units there are three square numbers, i.e. their roots are integers and are known, and they are 1, 4, 9, whose roots are 1, 2, 3. |
| − | + | :<math>\scriptstyle{\color{blue}{\sqrt{1}=1\quad\sqrt{4}=2\quad\sqrt{9}=3}}</math> | |
| − | :<math>\scriptstyle{\color{blue}{2\ | + | |style="text-align:right;"|דע כי במעלת האחדי' יש בה ג' מספרי' מרובעי' ר"ל ששרשם שלמי' וידועי' והם א' וד' וט' ששרשם א' ב' ג‫' |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| − | | | + | |The second rank, which is [the rank of] tens, does not have a square number at all, only together with units, because 10, 20, 30, 40, 50, 60, 70, 80, 90 none of them is a square number. Yet with the addition of units, it has six squares: like 16, whose root is 4; 25, whose root is 5; 36, whose root is 6; 49, whose root is 7; 64 whose root is 8; 81, whose root is 9. |
| − | : | + | |style="text-align:right;"|ובמעלה השנית שהי' עשרות [אין בה] מספר מרובע כלל רק עם חבור אחדי' כי י'כ'ל'מ'נ'ס'ע'פ'צ' אי גם אחד מהם מרובע אך עם חבור אחדי' יש בה ו' מרובעי' כמו י"ו שרשו ד' כ"ה שרשו ה' ל"ו שרשו ו' מ"ט שרשו ז' ס"ד שרשו ח' פ"א שרשו ט‫' |
| − | |style="text-align:right;"| | + | |- |
| + | |colspan="2" | | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{16}=4\quad\sqrt{25}=5\quad\sqrt{36}=6\quad\sqrt{49}=7\quad\sqrt{64}=8\quad\sqrt{81}=9}}</math> | ||
| + | |- | ||
| + | |Every odd rank, such as the hundreds, which is third; the tens of thousands, which is fifth; the seventh, the ninth, and the like endlessly, is analogous to the rank of units, because there are also three squares in them. | ||
| + | |style="text-align:right;"|וכל מעלה נפרד' כגו' המאו' שהי' השלישי' או הרבואו' שהם חמישית ושביעי' ותשיעי' וכן כלם דומי' דומי' למעל' האחדי' בשיש בם ג"כ ג' מרובעי‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | :As in the hundreds: 100, whose root is 10; 400, whose root is 20; 900, whose root is 30, and so on. |
| − | :<math>\scriptstyle{\color{blue}{ | + | :<math>\scriptstyle{\color{blue}{\sqrt{100}=10\quad\sqrt{400}=20\quad\sqrt{900}=30}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כגו' במאו' יש ק' ושרשו י' ת' ושרשו כ' ת'ת'ק' ושרשו ל' וכן כלם |
| + | |- | ||
| + | |The squares in the odd ranks are always [analogous to] 1, 4, 9 and their roots are [analogous to] 1, 2, 3 in the preceding rank to its immediate right. | ||
| + | |style="text-align:right;"|ולעול' א'ד'ט' מרבעי' במעלו' נפרדו' ושרשם א'ב'ג' מהמעלה שלפניה וימינה הקרובה לה | ||
|- | |- | ||
| − | | | + | |Every even rank is analogous to the tens. |
| − | + | |style="text-align:right;"|וכן כל מעלה זוגית דומ' לעשרו‫' | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | : | + | :As the thousands, in which there are six squares. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כגו' האלפי' יש בה ו' מרובעי‫' |
|- | |- | ||
| − | | | + | |As the squares in the tens are with addition of units, which is the closest rank to its right, so you always add to the even rank units from the preceding rank, which are added as units of the tens. |
| − | + | |style="text-align:right;"|וכמו שבעשרו' המרובעי' עם חבור אחדי' מה שהיא המעל' הקרובה לימינ לפניה כן תחבר לעולם עם המעל' הזוגיי' אחדי' מהמעלה שלפניה במספר שתצטרך להוסיף אחדי' ע' העשרו‫' | |
| − | |||
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | : | + | :As in the thousands: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כגו' באלפי‫' |
|- | |- | ||
| | | | ||
| − | : | + | :One thousand and 600, which is analogous to 16, whose root is 40, which is analogous to 4. |
| − | :<math>\scriptstyle{\color{blue}{ | + | :<math>\scriptstyle{\color{blue}{\sqrt{1600}=40\quad\sqrt{16}=4}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|יש אלף ות"ר הדומ' לי"ו ושרשו ת'מ' והו' דומ' לד‫' |
|- | |- | ||
| | | | ||
| − | : | + | :Two thousand and 500, which is analogous to 25, whose root is 50, which is analogous to 5. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{\sqrt{2500}=50\quad\sqrt{25}=5}}</math> |
| + | |style="text-align:right;"|אלפים ות"ק הדומ' לכ"ה ושרשו נ' הדומ' לה‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | :3 thousand and 600, which is analogous to 36, whose root is 60, which is analogous to 6. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{\sqrt{3600}=60\quad\sqrt{36}=6}}</math> |
| + | |style="text-align:right;"|ג' אלפי' ות"ר הדומ' לל"ו ושרשו ס' הדומ' לו‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | :4 thousand and 900, which is analogous to 49, whose root is 70, which is analogous to 7. |
| − | :<math>\scriptstyle{\color{blue}{ | + | :<math>\scriptstyle{\color{blue}{\sqrt{4900}=70\quad\sqrt{49}=7}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וד' אלפים תת"ק הדומ' למ"ט ושרשו ע' הדומ' לז‫' |
|- | |- | ||
| | | | ||
| − | : | + | :6 thousand and 400, which is analogous to 64, whose root is 80, which is analogous to 8. |
| − | :<math>\scriptstyle{\color{blue}{ | + | :<math>\scriptstyle{\color{blue}{\sqrt{6400}=80\quad\sqrt{64}=8}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וו' אלפי' ות' הדומ' לס"ד ושרשו פ' הדומ' לח‫' |
|- | |- | ||
| | | | ||
| − | : | + | :8 thousand and 100, which is analogous to 81, whose root is 90, which is analogous to 9. |
| − | :<math>\scriptstyle{\color{blue}{ | + | :<math>\scriptstyle{\color{blue}{\sqrt{8100}=90\quad\sqrt{81}=9}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וח' אלפי' ק' הדומ' לפ"א ושרשו צ' הדומ' לט‫' |
| + | |- | ||
| + | |Likewise for every even rank. | ||
| + | |style="text-align:right;"|וכן כל מעל' זוגיית | ||
| + | |- | ||
| + | |This is the rule: every square of an odd rank is [analogous to] 1, 4, 9, whose roots are 1, 2, 3; and the squares of every even rank are [analogous to] 16, 25, 36, 49, 64, 81, whose roots are 4, 5, 6, 7, 8, 9, but the rank of the roots is never the closest to it. | ||
| + | |style="text-align:right;"|וזה הכלל כל מרובע של מעלה נפרדת הו' א' ד' ט' ושרשם א' ב' ג' וכל מעל' זוגיי' שרש מרובעיה ו"א ה"ב ו"ג ט"ד ד"ו א"ח ושרשם ד' ה' ו' ז' ח' ט' ואבל אי' מעלת השרשי' לעול' הסמוכה לה | ||
|- | |- | ||
| − | | | + | |This is how you know the positional rank of the root: always look for an odd rank to shift the root from the square back to it as the number of ranks it is away from the units. |
| − | : | + | |style="text-align:right;"|וכה תדע מעלת מקו' השרש לעולם ראה במספרי' מעל' נפרדת להשיב השרש רחוק מהמרובע אחורני' מספר מעלו' כמספר מעלו' שירחק מהאחדי‫' |
| − | |||
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | : | + | *Example: we find 4 in the ninth rank, like this: |
| − | : | + | |style="text-align:right;"|והמשל מצאנו ד' במעל' תשיעי' כזה |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | : | + | ::{|class="wikitable" style="color:blue; text-align:center;" |
| − | : | ||
| − | |||
|- | |- | ||
| | | | ||
| − | + | {|style="margin-left: auto; margin-right: 0px;" | |
| − | + | |- | |
| − | |style=" | + | |4||0||0||0||0||0||0||0||0 |
|- | |- | ||
| + | | || || || ||2|| || || || | ||
| + | |} | ||
| + | |} | ||
| | | | ||
| − | |style="text-align: | + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" |
|- | |- | ||
| | | | ||
| − | |style=" | + | {|style="margin-left: auto; margin-right: 0px;" |
| + | |- | ||
| + | |ד||0||0||0||0||0||0||0||0 | ||
| + | |- | ||
| + | | || || || ||ב|| || || || | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :We know that this number is square, because it is in an odd rank, so it is analogous to the units; in the units 4 is a square and its root is 2, so its root is also 2. |
| + | |style="text-align:right;"|הנה ידענו כי זה המספר מרובע כי הו' מעלה נפרד' ודומ' לאחדי' ובאחדי' ד' הו' מרובע ושרשו הו' ב' א"כ גם זה שרשו ב‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :The position of the 2 should be in the middle between the rank of the units and the rank of the square, meaning the fifth [rank]. |
| + | |style="text-align:right;"|ומקומה של הב' ראויה להיות ממוצעת בין מעלת האחדי' ומעלת המרובע והיינו בחמשית | ||
| + | |- | ||
| + | |Also for an even rank, since you always have to add the units of the preceding rank to the square and you have to shift the even rank back and consider it as tens in an odd rank, do as mentioned above and write the root in the middle. | ||
| + | |style="text-align:right;"|וכן מספרי' הזוגו' מאחר שצריך לך לעול' לחבר עם המרובע אחדי' מהמעלה שלפניו וצריך להשיב מעלת הזוג אחורנית לחשבה בעשרו' ויהיו שם במעלה נפרדת עשה ג"כ כנ"ל ושים השרש באמצע | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :As if you say: we find 36 in the eighth rank, which is even, and it is analogous to 36, which is a square in the tens and its root is 6, so its root is also 6 and the 6 must be written between the beginning of the square number and the units, in the middle, that is, in the fourth rank, which is 3 ranks away from the units, and so is its distance from the [seventh] rank, where the square number begins. |
| + | |style="text-align:right;"|כאלו תאמ' מצאנו ו'ג' במעל' ח' והי' זוגיי' ודומה לל"ו שבעשרו' שהו' מרובע ושרשו ו' א"כ גם זה שרשו ו' וצרי' להשים הו' בין תחלת המספר המרובע ובין האחדי' באמצע דהיינו במעל' ד' שהו' רחוק מהאחדי' ג' מעלו' וכן הו' רחוק מהמעל' הה' אשר שם החל מספר המרובע | ||
|- | |- | ||
| | | | ||
| − | |style="text-align: | + | ::{|class="wikitable" style="color:blue; text-align:center;" |
|- | |- | ||
| | | | ||
| − | + | {|style="margin-left: auto; margin-right: 0px;" | |
| − | = | ||
| − | |||
|- | |- | ||
| − | | | + | |3||6||0||0||0||0||0||0 |
| − | | | ||
|- | |- | ||
| − | | | + | | || || ||6|| || || || |
| − | |style="text-align: | + | |} |
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
|- | |- | ||
| − | | | + | | |
| − | |style=" | + | {|style="margin-left: auto; margin-right: 0px;" |
|- | |- | ||
| − | | | + | |ג||ו||0||0||0||0||0||0 |
| − | | | ||
|- | |- | ||
| − | | | + | | || || ||ו|| || || || |
| − | | | + | |} |
|- | |- | ||
| − | | | + | |} |
| − | |||
|- | |- | ||
| − | | | + | |Here is the procedure for knowing the approximate or real root: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והילך מעשה ידיע' השרש הקרו' או האמתי |
|- | |- | ||
| − | | | + | |Write the number whose root is required in one row, according to its ranks. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כתו' המספר הדרוש שרשו בטור אחד כפי מעלותיו |
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | *Below is an example of 5 ranks as follows: |
| + | |style="text-align:right;"|המשל בזה שמטהו ה' מעלו' כזה | ||
|- | |- | ||
| − | | | + | | |
| − | | | + | ::{|class="wikitable" style="color:blue; text-align:center;" |
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | + | {|style="margin-left: auto; margin-right: 0px;" | |
| − | = | ||
| − | |||
|- | |- | ||
| − | | | + | | ||0||0||3||2 |
| − | | | ||
|- | |- | ||
| − | | | + | |0||1||4|| || |
| − | | | ||
|- | |- | ||
| − | | | + | |1|| || || || |
| − | | | ||
|- | |- | ||
| − | | | + | |5||4||3||2||1 |
| − | | | ||
|- | |- | ||
| − | | | + | | || ||2||3||3 |
| − | | | ||
|- | |- | ||
| − | | | + | | || ||4||6|| |
| − | | | + | |} |
| − | | | ||
| − | | | ||
| − | | | ||
|- | |- | ||
| | | | ||
| − | + | {|style="margin-left: auto; margin-right: 0px;" | |
| − | + | |- | |
| − | | | + | |4||0||0|| || |
|- | |- | ||
| − | | | + | |1||2||9||0|| |
| − | | | ||
|- | |- | ||
| − | | | + | | ||1||3||8||9 |
| − | | | + | |} |
|- | |- | ||
| − | | | + | | |
| − | |style=" | + | {|style="margin-left: auto; margin-right: 0px;" |
|- | |- | ||
| − | | | + | |5||4||2||8||9 |
| − | | | ||
|- | |- | ||
| − | | | + | | || || ||3||2 |
| − | | | + | |} |
|- | |- | ||
| | | | ||
| − | + | {|style="margin-left: auto; margin-right: 0px;" | |
| − | |||
| − | |||
| − | |style=" | ||
|- | |- | ||
| − | | | + | |5||4||3||2||1 |
| − | | | + | |} |
|- | |- | ||
| − | | | + | |} |
| − | |style="text-align: | + | | |
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
|- | |- | ||
| − | | | + | | |
| − | |style=" | + | {|style="margin-left: auto; margin-right: 0px;" |
|- | |- | ||
| − | | | + | | ||0||0||ג||ב |
| − | | | ||
|- | |- | ||
| − | | | + | |0||א||ד|| || |
| − | | | ||
|- | |- | ||
| − | | | + | |א|| || || || |
| − | | | ||
|- | |- | ||
| − | | | + | |ה||ד||ג||ב||א |
| − | | | ||
|- | |- | ||
| − | | | + | | || ||ב||ג||ג |
| − | | | ||
|- | |- | ||
| − | | | + | | || ||ד||ו|| |
| − | | | + | |} |
|- | |- | ||
| − | | | + | | |
| − | |style=" | + | {|style="margin-left: auto; margin-right: 0px;" |
|- | |- | ||
| − | | | + | |ד||0||0|| || |
| − | | | ||
|- | |- | ||
| − | | | + | |א||ב||ט||0|| |
| − | | | ||
|- | |- | ||
| − | | | + | | ||א||ג||ח||ט |
| − | | | + | |} |
|- | |- | ||
| | | | ||
| − | |style=" | + | {|style="margin-left: auto; margin-right: 0px;" |
| + | |- | ||
| + | |ה||ד||ב||ח||ט | ||
| + | |- | ||
| + | | || || ||ג||ב | ||
| + | |} | ||
|- | |- | ||
| | | | ||
| − | |style=" | + | {|style="margin-left: auto; margin-right: 0px;" |
| − | + | |- | |
| + | |ה||ד||ג||ב||א | ||
| + | |} | ||
|- | |- | ||
| − | | | + | |} |
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :You know from the above that this number [is in] an odd [rank] and it is analogous to the units, i.e. the 5. The closest root to it is 2, which is the root of 4, which is closest to it, preceding it. |
| + | |style="text-align:right;"|והנה ידעת מהנ"ל שזה המספר נפרד ודומ' לאחדי' ר"ל הה' ושרש היותר קרו' בה הו' ב' שהו' שרש ד' הקרוב לה לפניו | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :The place of this 2 as above is in the third rank beneath the 3. |
| + | |style="text-align:right;"|ומקו' הב' כנ"ל הו' במעל' ג' תחת הג‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Now, see how many ranks there are from the units to the place of the 2, which is your root, and start writing zeros from there, as the number of these ranks. |
| + | |style="text-align:right;"|ראה מעתה כמה מעלו' עברו מהאחדי' עד מקו' הב' שהי' שרשך ושם החל לכתו' גלגלי' כמספר המעלו' ההם | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :As, if you say: from the units to the 2 there are 2 ranks, so we write two zeros beneath the line, starting from the 2 itself and going to the left. |
| + | |style="text-align:right;"|כאלו תאמ' מהאחדי' עד הב' יש ב' מעלו' לכן נכתו' תחת הקו ב' גלגלי' מתחילי' תחת הב' בעצמ' והולכי' לשמאל | ||
|- | |- | ||
| − | | | + | | |
| − | + | :Then, we write the product of 2 by 2 there and this is 4. | |
| + | |style="text-align:right;"|אח"כ נכתו' העול' מכפל ב' על ב' שם והו' ד‫' | ||
|- | |- | ||
| | | | ||
| − | + | :Subtract the 4 from the 5 of the required number; 1 remains. | |
| − | + | :<math>\scriptstyle{\color{blue}{5-4=1}}</math> | |
| + | |style="text-align:right;"|אח"כ גרע הד' מהה' של מספר הדרוש וישאר א‫' | ||
| + | |- | ||
| | | | ||
| + | :Write 1 above the 5 and strike out the 5, because it is not needed any more. | ||
| + | |style="text-align:right;"|וכן כתו' א' ממעל לה' והעבר קו סביב הה' כי אי' בה צורך עוד | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Now, you should to know the root that is closer than the 2: |
| − | + | |style="text-align:right;"|ומעתה נשאר לדעת השרש היותר קרוב מהב‫' | |
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | + | :You should take a number to add to the root, so that you add double that number to twice the root, which is 2 in our example, in the rank of hundreds, as well as the product of that number by itself. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וזה יצטרך לקחת לכל מה מספר שתוסיף על השרש כפל אותו מספר על פעמים זה השרש שהו' הב' במשלינו במעל' המאות וגם כפל המספר ההו' על עצמו |
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | :The proof of this is that we assume a square number of 4 tens of thousands, so its root is 2 hundred, as you have seen, and its root is one side of the square itself. Because a length of 2 hundred cubits by a width of 2 hundred cubits is 4 tens of thousands of cubits by cubit. |
| + | |style="text-align:right;"|ומופת זה כי אנחנו הנחנו מספר מרובע של ד' רבואו' א"כ שרשו ב' מאו' כמ' שראית ושרשו הוא מספר הצלע האחת מהמרובע בעצמ' כי ב' מאו' אמ' ארך על ב' מאו' אמ' רחב ימצא בם ד' רבואו' אמו' של אמה על אמ‫' | ||
|- | |- | ||
| | | | ||
| − | + | :Suppose we add thirty to the root of this square, so one side of this square is 230. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ונניח שנוסיף על שרש זה המרובע <s>ג'</s> שלשי' ויהיה צלע אחד של המרובע ר"ל |
|- | |- | ||
| | | | ||
| − | : | + | :We find that when you put 30 strips, each of which is two hundred cubits long and 1 cubit wide, that is, one strip of two hundred by 30, and you add them to one side of this square - as, if you say to its east - then, its length exceeds its width - its length is 230 and its width is 200. |
| − | + | |style="text-align:right;"|נמצא שתשים ל' רצועו' כל אחת של מאתים אמ' ארך וא' אמ' רחב דהיינו רצועה אחת של מאתים על ל' ותחברנה בצלע אחד מהמרובע כאלו תאמ' למזרחו ויהיה המרובע אז ארכו יתר על רחבו ארכו ר"ל ורחבו ר‫' | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | : | + | :Then, you add another strip of 200 by 30 to the south of the square, so its width is also 230. |
| − | + | |style="text-align:right;"|אח"כ הוספת עוד רצוע' <s>ל'</s> של ר' על ל' אז לדרומו של המרובע ויהיה רחבו ג"כ ר"ל | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | :Therefore, you already added to this [square] a product of 30 by 200 - once to the east and once to the south. |
| + | |style="text-align:right;"|וא"כ כבר הוספת על זה השרש כפל ל' על ר' פעמי' פעם א' המזרח ופעם א' הדרום | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :This is a product of 30 by 400. |
| + | |style="text-align:right;"|<s>ועו</s> והו' הו' כפל ל' על ת‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :There is still a "remote corner" missing in this rectangle, and it is the southeastern corner, which is not filled until after you put there a square of 30 by 30. Then, the rectangle becomes an equilateral square, whose root is 230. |
| + | |style="text-align:right;"|ועדיין חסרה קרן זוית בזה המרובע והי' הקרן דרומי' מזרחי' אשר לא תתמלא רק אחר שומך שם מרובע ל' על ל' ואז ישוב מרובע שוה הצלעו' שרשו ר"ל | ||
| + | |- | ||
| + | |Illegible | ||
| + | |style="text-align:right;"|‫[...] והיוצא מזה [...] שתכפול מה שתרצה להוסיף עליו על עצמו אח"כ על ב' פעמי [...] לעשו' שיהא בא צריך להוסיף עליו כפל ד' על עצמו שהו' י"ו [...] פעמי [...] גם כפל ד' ל"ד שהו' ב' פעמי' י"ז | ||
| + | |- | ||
| + | |Illegible | ||
| + | |style="text-align:right;"|ונשוב לכונתינו ונאמ' אחרי שמצאת [...] וכתבתם [..] על מקומ' גם חסרונ' מרובעה של הב' מה"ה ונשאר א' שוב מעתה הוסיף על השרש מהמספר הנשאר והו' א' ב' ג' ד' א' ככל מה שתוכל וזה הדרך תלך בו | ||
| + | |- | ||
| + | |Illegible | ||
| + | |style="text-align:right;"|קח השרש שמצאת ב' פעמים ר"ל כפול אותו עם ב' אם הו' ג' כתו' ו' ואם ח' ו'א' ובדמיוננו הו' ב' לכן כת'[...] במעלתו ד‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Now, divide the upper number by the 4 and see how many times 4 is in the last 1 with the 4 that precedes it, when you shift it backwards and they become 14: |
| + | |style="text-align:right;"|וחלק מעתה המספר העליון על הד' וראה כמ' פעמי' ימצא ד' בא' האחרונ' עם הד' שלפניה כשתשיבנה אחורני' ויהיו י"ד | ||
|- | |- | ||
| | | | ||
| − | + | :It is found 3 times, but you need to see if it is enough to fill the "remote corner", which is 9 and it is the product of 30 by 30. | |
| − | + | |style="text-align:right;"|הוי או' ג' פעמי' ימצא וצריך לראו' אם ימצא ג"כ למלאת הקרן זוי' והו' ט' <s>וו</s> שהו' כפל 0"ג על 0"ג | |
| − | + | |- | |
| | | | ||
| + | :If it was not enough, I would take only 2, but in our example we can take 3. | ||
| + | |style="text-align:right;"|כי אם לא היה אפשר לא הייתי לוקח רק ב' והנה בדמיוננו נוכל לקח[ת] ג‫' | ||
|- | |- | ||
| − | |||
| | | | ||
| + | :We write 3 before the 2, to its right. | ||
| + | |style="text-align:right;"|ונכתו' מעת' ג' קדם הב' לימינ‫' | ||
|- | |- | ||
| | | | ||
| − | + | :Again do as above, like this: | |
| − | + | |style="text-align:right;"|<s>ומ</s> ושוב לעשו' כנ"ל והו' זה | |
| − | |||
| − | : | ||
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | : | + | :See how many ranks there are from the units to the 3; there is one. |
| − | + | |style="text-align:right;"|ראה כמ' מעלו' מהאחדי' עד הג' והנה הי' אחת | |
| − | |style=" | ||
|- | |- | ||
| | | | ||
| − | : | + | :So, write one zero beneath the line in the second rank, i.e. beneath that 3. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|לכן כתו' תחת הקו במעלה ב' דהיינו תחת הג' ההי' גלגל אחד |
|- | |- | ||
| | | | ||
| − | : | + | :Then, multiply 3 by itself; the result is 9. |
| − | + | :<math>\scriptstyle{\color{blue}{3^2=9}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אח"כ כפול ג' על עצמו ויצא ט‫' |
|- | |- | ||
| | | | ||
| − | : | + | :Write it in its place beneath the line, to the left of the single zero you wrote below the 3. |
| − | + | |style="text-align:right;"|כתבהו במקומו תחת הקו לשמאל הגלגל היחיד שעשית תחת הג‫' | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| − | |||
| | | | ||
| + | :Multiply again the 3 by the 4, which is twice the root; the result is 12. | ||
| + | :<math>\scriptstyle{\color{blue}{3\sdot\left(2\sdot2\right)=3\sdot4=12}}</math> | ||
| + | |style="text-align:right;"|שוב כפול הג' על <s>בב'</s> הד' שיהי' ב' פעמי' השרש ויעלה ב"א | ||
|- | |- | ||
| | | | ||
| − | + | :Write it in its place after the 9, beneath the line. Because the 3 is of the rank of tens and the 4 is of the rank of hundreds and the product of tens by hundreds is thousands. So, start writing the 2 beneath the rank of thousands and the 1 beneath the tens of thousands. | |
| − | + | |style="text-align:right;"|וכתבם במקומ' אחרי הט' תחת הקו כי הג' הי' ממעל' העשרו' והד' מהמאו' וכפל עשרו' במאו' יעלו אלפי' לכן תחל לכתו' הב' תחת מעלת האלפי' והא' תחת הרבואו‫' | |
| − | + | |- | |
| − | : | + | | |
| − | + | :Then, subtract it from the upper number, each from its corresponding: | |
| − | : | + | |style="text-align:right;"|אח"כ גרע זה מהמספר העליון כל מין ממינו |
| − | |style="text-align:right;"| | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :Meaning, the 1 from 1 - nothing remains; the 2 from 4 - 2 remains. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{1-1=0}}</math> |
| + | :<math>\scriptstyle{\color{blue}{4-2=2}}</math> | ||
| + | |style="text-align:right;"|דהיינו הא' מן הא' ולא ישאר דבר והב' מהד' ישאר ב‫' | ||
|- | |- | ||
| | | | ||
| − | :: | + | :Since we cannot subtract the 9 from 3, we shift 1 backwards and write 1. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{2-1=1}}</math> |
| + | |style="text-align:right;"|ולפי שלא נוכל לקחת אז הט' מהג' לכן נשיב א' אחורני' ונכתו' א‫' | ||
|- | |- | ||
| | | | ||
| − | :: | + | :We subtract the 9 from the 1 that is shifted backwards to the 3, so they are 13; 4 remains. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{\left(10+3\right)-9=13-9=4}}</math> |
| + | |style="text-align:right;"|ונקח הט' מהא' המושבת אחורני' על הג' שהם י"ג וישארו ד‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | :We write 4 and we write all above the upper line each in its rank as you see in our diagram. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכן נכתו' ד' ונכתו' זה למעלה מהטור העליון איש איש על מעלתו כמו שתראה בדמיונינו זה |
|- | |- | ||
| | | | ||
| − | : | + | :Strike out the 4, 3 and 1, because they are not needed. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והעבר קו על ג"ד ועל א' כי אי' בם צרך |
|- | |- | ||
| | | | ||
| − | : | + | :You find the approximate root 230. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והנה מצאת שרש הקרו' ר"ל |
|- | |- | ||
| − | |||
| | | | ||
| + | :You want to increase the square and root further, because there are more digits of the original required number, since you are still left with 1421. | ||
| + | |style="text-align:right;"|עו' תרצה להגדיל המרובע והשרש כי יש עוד מספר רב מן המספר הראשון הדרוש כי נשאר לך עדיין א' ב' ד' א' <s>לכן חלקהו על הג' ותראה כמה פעמי' ימצא ג' ב</s> | ||
|- | |- | ||
| | | | ||
| − | + | :Multiply the 3 also by 2; it is 6. | |
| − | + | :<math>\scriptstyle{\color{blue}{2\sdot3=6}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ושוב מעתה לכפול גם הג' בב' ויהיה ו‫' |
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :Write it beneath the 3. |
| − | + | |style="text-align:right;"|כתבה תחת הג‫' | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | : | + | :Now, divide the number remaining from the required by double the whole root, which is 46, meaning 460, because the root is 230. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{2\sdot230=460}}</math> |
| + | |style="text-align:right;"|ומעתה חלק המספר הנותר מהדרוש על כפל כל השרש והו' ו'ד' דהיינו ת"ס כי השרש הו' ר"ל | ||
|- | |- | ||
| | | | ||
| − | : | + | :See how many times 4 is in 14; it is 3 times in it. |
| − | + | |style="text-align:right;"|וראה כמה פעמי' ימצא ד' בי"ד והנהו ג' פעמי‫' | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| − | |||
| | | | ||
| + | :Write 3 before the 23 of the root, beneath the 1. | ||
| + | |style="text-align:right;"|כתו' ג' לפני הג'ב' של השרש ותחת הא‫' | ||
|- | |- | ||
| | | | ||
| − | + | :Multiply 3 by itself; it is 9. | |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{3^2=9}}</math> |
| + | |style="text-align:right;"|וכפול מעתה ג' על עצמו ויהיה ט‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | :Write it beneath the line and beneath the 3 of the units, in order to complete the "remote corner", and since the 3 is not at all far from the rank of units, there is no need to write a zero. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כתבה תחת הקו ותחת הג' האחדית [ויהיה למלא הקרן זוית] ולפי שהג' אינ' רחוקה דבר ממעלת האחדי' ע"כ <sup>אין</sup> צרי' לשים שום גלגל |
|- | |- | ||
| | | | ||
| − | : | + | :Multiply the 3 also by twice the root, which is 46; you find 138. |
| − | + | :<math>\scriptstyle{\color{blue}{3\sdot\left(2\sdot23\right)=3\sdot46=138}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|עוד כפול הג' בשרש ב' פעמי' שהם ו'ד' ותמצא ח'ג'א‫' |
|- | |- | ||
| | | | ||
| − | : | + | :Write it in its place, meaning, start from the lower 9. |
| − | + | |style="text-align:right;"|כתבם על מקומ' דהיינו שתתחיל אצל הט' למטה | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| − | |||
| | | | ||
| + | :The result of multiplying 3 by itself and by double the root is 1389. | ||
| + | |style="text-align:right;"|ויהיה המספר היוצא מכפל ג' על עצמו ועל השרש פעמים ט ח ג א | ||
|- | |- | ||
| | | | ||
| − | + | :Subtract this number from what you have left of the required number, which is 1421: | |
| − | + | :<math>\scriptstyle{\color{blue}{1421-1389}}</math> | |
| − | + | |style="text-align:right;"|וזה המספר גרע מאשר נשאר לך מהמספר הדרוש והו' א ב ד א | |
| − | |style="text-align:right;"| | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :Subtract 1 from 1; nothing remains. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{1-1=0}}</math> |
| + | |style="text-align:right;"|והנה חסר א' מא' לא ישאר כלום | ||
|- | |- | ||
| | | | ||
| − | : | + | :Subtract 3 from 4; 1 remains. |
| − | + | :<math>\scriptstyle{\color{blue}{4-3=1}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|עוד חסר ג' מד' ישאר א‫' |
|- | |- | ||
| | | | ||
| − | : | + | :Since we cannot subtract 8 from 2, we should shift this 1 backwards, so nothing remains in this rank also. |
| − | + | :<math>\scriptstyle{\color{blue}{1-1=0}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ולפי שלא נוכל לקחת ח' מב' צריך להשיב זו הא' אחורנית ולא ישאר כלו' בזאת המעל' ג"כ |
|- | |- | ||
| − | |||
| | | | ||
| + | :Subtract 8 from 12; 4 remains. | ||
| + | :<math>\scriptstyle{\color{blue}{12-8=4}}</math> | ||
| + | |style="text-align:right;"|חסר ח' מב'א' ר"ל מי"ב ישאר ד‫' | ||
|- | |- | ||
| | | | ||
| − | + | :We still need to shift 1 backwards from the 4; 3 remains. Write it down. | |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{4-1=3}}</math> |
| + | |style="text-align:right;"|ועו' צרי' להשי' אחורני' א' מהד' נשאר ג' וכן תכתו‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | :Subtract 9 from 11; 2 remains. |
| − | + | :<math>\scriptstyle{\color{blue}{11-9=2}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|עו' חסר ט' מא"א והו' י"א וישאר ב‫' |
|- | |- | ||
| | | | ||
| − | : | + | :Write it in its place and crossed out 1421 because it is no longer needed. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכתבה במקומ' והעבר קו על א"ב ד"א כי אי' בם צורך עוד |
|- | |- | ||
| | | | ||
| − | : | + | :So, the required approximate root of 54321 is 233. |
| − | + | :<math>\scriptstyle{\color{blue}{\sqrt{54321}\approx233}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ויהיה השרש הקרוב למספר אבגדה בדרוש גגב |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :The square of 233 is as the sum of all the squares of the roots you extracted, which is 54289 and the remainder is 32. |
| − | + | :<math>\scriptstyle{\color{blue}{233^2=54289}}</math> | |
| − | + | |style="text-align:right;"|ויהיה המרובע כמספר כל המרובעי' שהוצאת מהשרשי' מגגב אחרי התחברם והו' טחבדה והנשאר מהמספר הו ב"ג | |
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :If you add 32 to the square, you receive the required number. |
| + | |style="text-align:right;"|ואם תשוב לחבר ב"ג על המרובע יצא לך המספר הדרוש | ||
|- | |- | ||
| − | | | + | |I will give you various rules, the knowledge of which is necessary for the root extraction procedure. |
| − | + | |style="text-align:right;"|אך אמנם אתן לך כללי' מתחלפי' יש צורך בידיעתם במעשה השרשים | |
| − | |||
| − | |style="text-align:right;"| | ||
|- | |- | ||
| − | | | + | |The first rule: when you extract the first root from the last digit you have, if it is in an odd rank, or in the preceding to the last, if it is in an even rank, you should write that root in the middle rank, as I have shown you and take as much as you can. If you cannot take anything from from that rank, and you take from the [rank] that is second to it, write it as far from the place, from which you take the quotient, as the first root is far from the units. So, you should write a zero in the place that precedes the first root, and before this zero you should write the result of the division. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|הכלל הא' כשלקחת השרש הראשון מהמספר האחרון שבידי אם הו' מעלה נפרדת או מהקודם לאחרון אם היא מעלה זוגיית ראוי לכתו' השרש ההו' במדרגה האמצעי' כהראיתיך ותקח כל מה שתוכל ואם לא תוכל לקחת דבר מהמקו' ההוא הראשון ולקחת מהשני לו גם כן תכתבהו רחוק מהמקו' שתקח ממנו ראש החלוק כאש[ר] השרש הראשון רחוק מהאחדי' ולכן ראוי לכתו' גלגל במקו' שלפני השרש הראשו' וק[ודם] לזה הגלגל תכתו' היוצא מהחלוק |
|- | |- | ||
| − | | | + | |The second rule: when you divide again what remains of the number you have by double the root, do not take the last number from which you took it first, even if you see that it is possible to take from it, because when you take the root from it first, you already take from it everything that should [be taken from it]. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|הכלל הב' כשתשוב לחלק הנשאר מהמספר שבידך על כפל השרש לא תקח המספר האחרון שלקחת ממנו ראשונה ואף כי מהמספר [שאחריו] ואף כי תראה כי האפשר לקחת בו מפני שכשלוקח ממנו השרש ראשונה כבר לו[קח ממנו כל הראוי |
|- | |- | ||
| | | | ||
| − | + | ||
| − | + | == Division of sexagesimal fractions == | |
| | | | ||
| − | |||
|- | |- | ||
| − | | | + | |When you wish to divide a number that consists of degrees, minutes and seconds by another number, smaller or greater: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|‫<ref>Guenzburg 196r</ref><big>כאשר</big> תרצה לחלק חשבון מעלות ודקים ושניים על חשבון אחר יותר קטן או יותר גדול |
|- | |- | ||
| − | | | + | |Take the number of the bottom row, which is the number by which you divide the number of the upper row, and convert all into the lowest type of fractions in it. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|תקח חשבון הטור התחתון והוא החשבון אשר עליו מחלק חשבון הטור העליון ותשבר הכל אל מין השבר היותר קטן אשר בו |
|- | |- | ||
| − | | | + | |I.e. if the lowest fraction in it is seconds, convert all into seconds; if thirds, convert all into thirds and so on. |
| − | |style="text-align:right;"|ואם | + | |style="text-align:right;"|ר"ל שאם השבר היותר קטן אשר בו הוא שניים תעשה מהכל שניים ואם שלשיים תעשה מה מהכל שלשיים וכן על זה הדרך |
| + | |- | ||
| + | |Then, take the number of the top row, which is the number divided by the number of the bottom row, and convert all into the [type of] fractions, which is as far from the type of fractions, into which you converted the bottom [number], as the type you want to receive in the division is far from the degrees. | ||
| + | |style="text-align:right;"|ואחר כן קח חשבון הטור העליון והוא החשבון אשר יחולק על חשבון הטור התחתון וישבר אותו אל מן השברים שיהיה רחוק ממין השברים ששברת בו התחתון כמו שיהיה מרחק המין שתרצה שיצא לך בחלוקה מן המעלות | ||
| + | |- | ||
| + | |I.e. if you converted the number of the bottom row into seconds and you want to receive thirds in division, convert the number of the top row into fifths that are three ranks away from the seconds, as the distance of the thirds from the rank of the degrees; the result of division will be thirds. | ||
| + | |style="text-align:right;"|ר"ל שאם שברת חשבון הטור התחתון מן השניים ותרצה שיצא לך בחלוקה שלשיים הנה תשבר חשבון הטור העליון למין חמשיים שהוא רחוק <sup>מן</sup> השניים שלשה מדרגות כמו מרחק השלשיים מגדר המעלות וזה יצא בחלוקה שלשיים | ||
|- | |- | ||
| − | | | + | |If you want to be more precise, so that the result of division will be fourths, convert the top row into sixths that are four ranks away from the seconds, as the distance of the fourths from the rank of the degrees; then you will get fourths from the division. |
| − | + | |style="text-align:right;"|ואם תרצה לדקדק עוד שיצא בחלוקה רבעיים תשבר הטור העליון לששיים שהוא רחוק מהשניים ארבע מדרגות כמו מרחק הרבעיים מגדר המעלות ואז יצא לך בחלוקה רבעיים | |
| − | |||
| − | | | ||
|- | |- | ||
| − | | | + | |And so on this way. |
| − | + | |style="text-align:right;"|וכן על זה הדרך | |
| − | |||
| − | |style="text-align:right;"| | ||
|- | |- | ||
| − | | | + | |If you covert the bottom row into thirds and you want to be more precise, so that you will get fourths from the division, convert the number of the top row into sevenths that are four ranks away from the fractions of the bottom row, as the distance of the fourths from the rank of the degrees; then you will get fourths from the division. |
| − | + | |style="text-align:right;"|וכן אם שברת הטור התחתון לשלשיים ותרצה לדקדק עד שיצא לך בחלוקה רבעיים תשבר חשבון הטור העליון לשבעים שהוא רחוק משברי הטור התחתון ארבע מדרגות כמו מרחק הרביעיים מגדר המעלות ויצא לך בחלוק רביעיים | |
| − | |||
| − | |style="text-align:right;"| | ||
|- | |- | ||
| − | | | + | |If you want to be more precise, so that you will get fifths from the division, convert the number of the top row into eighths that are five ranks away from the fractions of the bottom row, as the distance of the fifths from the rank of the degrees; then you will get fifths from the division. |
| − | + | |style="text-align:right;"|ואם תרצה לדקדק עד שיצא לך בחלוקה חמשיים תשבר חשבון הטור העליון לשמניים שהוא רחוק משברי הטור התחתון חמש מדרגות כמו מרחק החמשיים מגדר המעלות ויצא לידך בחלוקה חמשיים | |
| − | |||
| − | | | ||
|- | |- | ||
| − | | | + | |And so on this way. |
| − | + | |style="text-align:right;"|וכן על זה הדרך | |
| − | |||
| − | |||
| − | |style="text-align:right;"| | ||
| − | |||
|- | |- | ||
| − | | | + | |This is enough for you. |
| − | + | |style="text-align:right;"|ודי לך בזה | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | |||
| + | == Extracting roots of sexagesimal fractions == | ||
| | | | ||
|- | |- | ||
| − | | | + | |If you wish to find a root of any number you have consisting of degrees, minutes and seconds as many as they may be. |
| − | + | |style="text-align:right;"|וכן אם תרצה למצוא שרש אי זה חשבון שיהיה בידך ממעלות הראשונים ושניים כפי מה שיהיה | |
| − | |||
| − | |||
| − | |style="text-align:right;"| | ||
| − | |||
|- | |- | ||
| − | | | + | |Know up to which [type of] fractions you wish the root to be approximated, i.e. fourths, or fifths, or sixths, or which ever type of fraction you wish the approximate root to be composed of and denominate the root by it. |
| − | + | |style="text-align:right;"|דע לך עד כמה שברים תרצה לדקדק שיצא בשרש ר"ל אם רבעיים או חמשיים או ששיים או אי זה מן שברים אשר תרצה לדקדק שיצא בשרש תקראהו מין השרש | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| − | | | + | |Then, convert the whole number you have into the type of fractions, which is as far from the type [of the fractions] of the root as the [type of the fractions of the] root is far from the degrees. |
| − | : | + | |style="text-align:right;"|ואחר זה תתיך כל החשבון אשר בידך אל מין השברים שיהיה רחוק ממין השרש כמו מרחק מן השרש מהמעלות |
| − | |style="text-align:right;"| | + | |- |
| + | |If the type [of the fractions] of the root is fourths, convert the number into eighths and the resulting root will be fourths. | ||
| + | |style="text-align:right;"|שאם מין השרש רביעיים תתיך חשבון לשמוניים ומה שיצא השרש יהיה רביעיים | ||
|- | |- | ||
| − | | | + | |If the type [of the fractions] of the root is fifths, convert the number into [tenths] and the resulting root will be fifths. |
| − | + | |style="text-align:right;"|ואם מין השרש חמישיים תתיך החשבון לרביעיים ומה שיצא בשרש זה יהיה חמשיים | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| − | | | + | |And so on this way. |
| − | + | |style="text-align:right;"|וכן על זה הדרך | |
| − | |||
| − | |||
| − | |style="text-align:right;"| | ||
| − | |||
|- | |- | ||
| − | | | + | |The rule of this method is that you multiply the type [of the fractions] of the root by its own name, one duplication: if its is seconds, convert them into fourths; if it is thirds, convert them into sixths; if it is fourths, convert them into eighths; if it is fifths, convert them into tenths. |
| − | : | + | |style="text-align:right;"|והכלל בזה הדרך שתכפול מן השרש בשלו המראה לו כפלה פשוטה שאם יהיה שניים תתיך לרביעיים ואם יהיה שלשיים תתיך לששיים ואם יהיה רביעיים תתיך לשמוניים ואם הוא חמישיים תתיך לעשריים |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | ==== | + | == Multiplication of sexagesimal fractions == |
| − | |||
| | | | ||
|- | |- | ||
| − | | | + | |When multiplying a number by a number, convert the top row into its lowest [type of] fraction. |
| − | + | |style="text-align:right;"|<big>אמנם</big> בכפילת חשבון עם חשבון תתיך הטור העליון אל מין השבר היותר קטון אשר בו | |
| − | |||
| − | |style="text-align:right;"|<big> | ||
|- | |- | ||
| − | | | + | |Convert also the bottom row into its lowest type of fraction. |
| − | + | |style="text-align:right;"|וכן הטור התחתון תתיך אל מין השבר היותר קטון אשר בו | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| − | | | + | |The result of multiplication will be of the type of fraction denominated by the sum of the types of both rows. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והיוצא בכפילה יהיה מין השבר יקרא בשם המחובר המחובר משום הטורים |
|- | |- | ||
| − | | | + | |I.e. if you multiply seconds by seconds, the result will be fourths. |
| − | + | |style="text-align:right;"|ר"ל שאם תכפול שניים על שניים יהיה היוצא רביעיי | |
| − | |||
| − | |style="text-align:right;"| | ||
|- | |- | ||
| − | | | + | |If seconds by thirds, the result will be fifths. |
| − | + | |style="text-align:right;"|‫<ref>Guenzburg 196v</ref>ואם שניים על שלשיים יהיה היוצא חמישיים | |
| − | |||
| − | |||
| − | |style="text-align:right;"| | ||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | == | + | == A Rule for Checking the Squares == |
| − | | | + | |
| + | |style="text-align:right;"|<big>כלל בבחינת המרובעים</big>‫<ref>Guenzburg om.</ref> | ||
|- | |- | ||
| − | | | + | |When you have a number and you wish to check if it is a square, you can check it with the square that precedes it, or with the square that follows it: |
| − | |style="text-align:right;" | + | |style="text-align:right;"|<big>כאשר</big> יהיה בידך מספר ותרצה לבחון אותו אם הוא מרובע תוכל לבחון אותו עם המרובע הקודם לו לפניו ועם המרובע הקודם לו לאחריו |
|- | |- | ||
| − | | | + | |First with the square that precedes it: |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{OliveGreen}{a^2+b}}</math> |
| + | |style="text-align:right;"|ראשונה עם המרובע הקודם לו לפניו | ||
|- | |- | ||
| − | | | + | |Consider the difference between the preceding square and the number you have. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|תחשוב המרחק שבין המרובע שעבר ובין המספר אשר בידך |
|- | |- | ||
| − | | | + | |Divide the difference by double the root of the preceding square, the least possible by as little as you can. |
| − | + | |style="text-align:right;"|חלק המרחק ההוא על כפל שרש המרובע שעבר פחת מן האפשר על המעט שתוכל | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| − | | | + | |Subtract the quotient [and] the square of the quotient from the difference. |
| − | + | :<math>\scriptstyle{\color{OliveGreen}{b-\frac{b}{2a}=\left(\frac{b}{2a}\right)^2}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|גרע מהמרחק ההוא היוצא מחלוקה וזה מרובע המספר היוצא מחלוקה |
|- | |- | ||
| − | | | + | |If the result of dividing the difference by double the preceding root plus the square of the quotient equals the difference you have, no more and no less, then the number is a square. Otherwise, you are wrong. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{OliveGreen}{\frac{b}{2a}+\left(\frac{b}{2a}\right)^2=b}}</math> |
| + | |style="text-align:right;"|ואם היוצא לך מחלק המרחק על כפל השרש שעבר עם מרובע המספר היוצא מחלוקה שוה אל המרחק אשר בידך לא פחות ולא יתר הנה המספר מרובע ואם לא הנה טעית‫<ref>שעבר: Guenzburg שנים</ref> | ||
|- | |- | ||
| − | | | + | |You can check it also with the square that follows it: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|תוכל לבחון גם כן עם המרובע הקודם לו לאחריו |
|- | |- | ||
| − | | | + | |Consider the difference between the number and the following square. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|תחשוב המרחק שבין מספר ובין המרובע העתיד |
|- | |- | ||
| − | | | + | |Divide the difference by double the following square, and give it as much possible by as little as you can. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|חלק המרחק ההוא על כפל שרש המרובע העתיד ונכון לו יותר על האפשר על המעט שנוכל |
|- | |- | ||
| − | | | + | |Subtract the difference and the square of the quotient from the quotient. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|גרע המרחק ומרובע המספר היוצא מחלוקה מהעולה בחלוק |
|- | |- | ||
| − | | | + | |If the result of division plus the square of the quotient equals the difference, then the number is a square. Otherwise, you are wrong. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואם העולה מהחלוקה עם מרובע המספר היוצא מחלוקה שוה למרחק הנה המספר מרובע ואם לא הנה טעות |
|- | |- | ||
| − | | | + | |Over and done |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|תם ונשלם |
|- | |- | ||
| − | | | + | |Praise be to the Lord of the world. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|תהלה לאל עולם |
| + | |- | ||
| + | |When the analogous square is less than the required number, we must take the greatest possible quotient so that the quotient is [equal to] the difference minus the square of the quotient. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\frac{b}{2a}=b-\left(\frac{b}{2a}\right)^2}}</math> | ||
| + | |style="text-align:right;"|כאשר יהיה המרובע הנמשל פחות מהמספר המבוקש צריך שנקח החלוק מספר היותר גדול שנוכל שיעלה בחלוקה המרחק כאשר יחוסר ממנו מרובע מה שיעלה בחלוק | ||
| + | |- | ||
| + | |When you add the product to the analogous square with the square of the quotient, it is the square closest to the required number. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{a^2+\left(\frac{b}{2a}\sdot2a\right)+\left(\frac{b}{2a}\right)^2}}</math> | ||
| + | |style="text-align:right;"|וכאשר תוסיף על המרובע הנמשל כמו הכפל עם מרובע מה שיעלה בחלוק הוא המרובע היותר קרוב על המספר המבוקש | ||
| + | |- | ||
| + | |When you add the quotient to the root of the analogous square, it is its root. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\sqrt{a^2+\left(\frac{b}{2a}\sdot2a\right)+\left(\frac{b}{2a}\right)^2}=a+\frac{b}{2a}}}</math> | ||
| + | |style="text-align:right;"|וכאשר תוסיף על שורש המרובע הנמשל מספר מה שיעלה בחלק הוא שרשו | ||
| + | |- | ||
| + | |When the required number is less than the analogous square, we must take the smallest possible quotient so that the quotient is [equal to] the sum of the difference and the square of the quotient. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\frac{b}{2a}=b+\left(\frac{b}{2a}\right)^2}}</math> | ||
| + | |style="text-align:right;"|וכאשר היה המספר המבוקש פחות מהמרובע הנמשל צריך שנקח החלוק מספר היותר קטן שנוכל שיעלה כמו המרחק מחובר עם מרובע מה שיעלה בחלוק | ||
| + | |- | ||
| + | |When you subtract the product from the analogous square [and add] the square of the quotient, it is the square closest to the required number. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{a^2-\left(\frac{b}{2a}\sdot2a\right)+\left(\frac{b}{2a}\right)^2}}</math> | ||
| + | |style="text-align:right;"|וכאשר תגרע מהמרובע הנמשל כמו הכפל כאשר יחובר ממנו מרובע מה שיעלה בחלוק הוא המרובע היותר קרוב אל המספר המבוקש | ||
| + | |- | ||
| + | |When you subtract the quotient from the root of the analogous square, it is its root. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\sqrt{a^2-\left(\frac{b}{2a}\sdot2a\right)+\left(\frac{b}{2a}\right)^2}=a-\frac{b}{2a}}}</math> | ||
| + | |style="text-align:right;"|וכאשר תגרע משרש הנמשל מספר מה שיעלה בחלוק הוא שרשו | ||
|- | |- | ||
| − | | | + | |The difference it the difference between the analogous square and the required number. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|המרחק הוא המרחק שבין המרובע הנמשל ובין המספר המבוקש |
|- | |- | ||
| − | | | + | |The product is the product of the quotient by double the root of the analogous square. |
| − | + | :<math>\scriptstyle{\color{OliveGreen}{\frac{b}{2a}\sdot2a}}</math> | |
| − | + | |style="text-align:right;"|הנכפל הוא מה שיעלה מכפלת מספר מה שיעלה בחלוק עם כפל שורש המרובע הנמשל | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| − | | | + | |Because, when there are two different squares, the ratio of the product of one root by the other to the first square is the same as the ratio of the second square to the product. |
| − | + | :<math>\scriptstyle{\color{OliveGreen}{\left(A\sdot B\right):A^2=B^2:\left(A\sdot B\right)}}</math> | |
| − | + | |style="text-align:right;"|כי יהיו שני מרובעים מתחלפים יחס המחובר מכפלת אחד מן השרשים על האחר אל המרובע הראשון כיחס המרובע השני אל המחובר | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | ||
| − | + | == <span style=color:green>MS London 7r-v</span> == | |
| − | |||
| | | | ||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |He said: the reasons of the division are two: |
| − | :: | + | |style="text-align:right;"|אמר טעם החלוק הם שניים |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :1) We multiply the result of the division according to the multiplication method by the bottom row, which is the divisor, then we add the remainder of the division to the product, if there is anything left. If the sum is equal to the upper row, which is the dividend, we calculated correctly. |
| + | |style="text-align:right;"|א שנכפול בדרך הכפל מה שיצא בחלוק על הטור השפל שמחלקים עליו ונחבר עם העולה מה שנשאר לחלק אם נשאר דבר ואם המחובר שוה למספר הטור המחולק עשינו נכונה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :2) We multiply the scales of the result of division by the scales of the bottom row; we take the result. If there is anything left, we add it to it. If it equals the scales of the upper row, our calculation is correct. |
| + | |style="text-align:right;"|הב' שנכפול מאזני מה שעלה בחלוק עם מאזני הטור השפל ונקח ממנו העולה ואם נשאר דבר לחלק נחבריהו עמו ואם הוא שוה למאזני הטור העליון חשבונינו אמת | ||
|- | |- | ||
| − | | | + | |The reason for the first scales is that the dividend number has as many times the divisor as the result of division, which is a number that counts the divisor in the greater number, which is the dividend. Therefore, [the product of] this by that is the dividend. |
| − | + | |style="text-align:right;"|והטעם למאזנים הראשונים כי בחשבון המחולק מדמיוני החשבון שמחלקים עליו כשיעור אחד מה שיעלה בחלוק הוא מספר מה שימנה המספר שמחלקים עליו למספר גדול המחולק לזה ככה זה על זה ויצא המחולק | |
| − | |||
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | : | + | === Chapter on Summation === |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<big>שער</big> המחברת |
| + | |- | ||
| + | |To know how much is the sum of numbers from a known number to the last number. | ||
| + | |style="text-align:right;"|לדעת כמה המחובר מהמספרי' ממספר ידוע עד סוף מספר | ||
|- | |- | ||
| | | | ||
| − | : | + | *As if you say: we wish to know the sum from one to ten, as 1 with 2 - it is 3; 3 with 4 - it is 7; and you want to know easily: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כאלו תאמר רצינו לדעת המחוברים מאחד עד עשרה כמו א' עם ב' הם ג' ג' עם ד' הם ז' ותרצה לדעת בקצור |
|- | |- | ||
| − | | | + | | |
| − | + | :If their number is even, as ten: we add 1 to it. Take a half of ten, which is five, and multiply the five by 11 and this is the last number [= the sum]. | |
| + | :<math>\scriptstyle{\color{blue}{\sum_{i=1}^{10} i=\left(10+1\right)\sdot\left(\frac{1}{2}\sdot10\right)=11\sdot5}}</math> | ||
| + | |style="text-align:right;"|אם הם זוג כמו עשרה ונוסיף עליו א' ותקח חצי העשר שהם חמשה וכפול החמשה על הי"א והוא המספר האחרון | ||
|- | |- | ||
| | | | ||
| − | + | :If [their number] is even, as from one to seven: add one to seven; it is 8. Take half of this number; it is 4. Multiply 4 by 7 and this is the required. | |
| − | |style="text-align:right;"|ואם | + | :<math>\scriptstyle{\color{blue}{\sum_{i=1}^7 i=\left[\frac{1}{2}\sdot\left(7+1\right)\right]\sdot7=\left(\frac{1}{2}\sdot8\right)\sdot7=4\sdot7}}</math> |
| + | |style="text-align:right;"|ואם נפרד כמו מאחד עד שבע תוסיף על ז' אחד ויהיו ח' ותקח חצי המספר והם ד' וכפול הד' על הז' והוא המכוון | ||
| + | |- | ||
| + | |Do this with any number, small of large, even or odd. | ||
| + | |style="text-align:right;"|וכן תעשה בכל מספר קטן או גדול זוג א[ו] נפרד | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *If you wish to find an unknown term, as if you say: the ratio of 4 to 6 is the same as the ratio of 8 to the unknown and you wish to find [the unknown term] |
| + | :<math>\scriptstyle{\color{blue}{4:6=8:a}}</math> | ||
| + | |style="text-align:right;"|אם תרצה לדעת ערך נעלם כמו שתאמר ערך ד' אל ו' כערך ח' אל הנעלם ותרצה לדעת | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :[Multiply] the means [by each other], 6 by 8; it is 48. |
| + | |style="text-align:right;"|האמצעיים ו' על ח' יהיו מ"ח | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Divide [it by] the first number, which is 4; you receive [12], which is the unknown. |
| + | :<math>\scriptstyle{\color{blue}{a=\frac{6\sdot8}{4}=\frac{48}{4}}}</math> | ||
| + | |style="text-align:right;"|ותחלק המספר הראשון שהוא ד' על המ"ח ויעלה לך חלק מ"ח והוא הנעלם | ||
| + | |- | ||
| + | |Abraham said: I have found another way: add to the square of the last number its root, then take half of the result; that is the sum. This way helps to understand the inverse question. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\sum_{i=1}^n i=\frac{1}{2}\left(n^2+n\right)}}</math> | ||
| + | |style="text-align:right;"|[[ספר_המספר_/_אברהם_אבן_עזרא#Abraham said|<span style=color:blue>'''אמר אברהם'''</span>]] מצאתי דרך אחר שהמוסיף על מרובע סוף החשבון שרשו ולוקח חצי בעולה שם ימצא המחובר והדרך הזה יורה להבין הפך השאלה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *Example: one asks: I added up numbers and the result is 465; how much is the last number? |
| + | |style="text-align:right;"|דמיון שאל שואל חברתי מספרים עלו תס"ה כמה יהיה סוף החשבון | ||
| + | |- | ||
| + | |This is the rule: take the sum, always double the sum, then take the root of the square, i.e. the preceding square, and check it: if between the square and the double remains the root no more and no less, know that the root is the required. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\sum_{i=1}^{n} i=a\longrightarrow n=2a-n^2}}</math> | ||
| + | |style="text-align:right;"|כלל זה תקח בידך היוצא [...] כפול לעולם החשבון המחובר והשורש תקח מן המרובע כלו' פי' הנכפל שעבר ובחן אותו כ"א נשאר בין המרובע ובין הנכפל כמו שורש בלי תוספת ומגרעת תדע כי החשבון והשורש הוא המבוקש | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :We double 465; the result is 930. We know that the preceding square is 9 hundred and its root is 30. Between the square and the double there is none but 30, so you understand that the calculation is correct and the required is the root. |
| + | :<math>\scriptstyle{\color{blue}{n=\left(2\sdot465\right)-30^2=930-900=30}}</math> | ||
| + | |style="text-align:right;"|והנה כפלנו תס"ה על(ה) תתק"ל ידענו כי מרובע שעבר הוא ט מאות ושרשו ל' שהם המספרי' המרובעי' ואין בין המרובע והנכפל כ"א ל' ועל כן תבין כי נכון מה שחשב והמבוקש ל‫' | ||
|- | |- | ||
| | | | ||
| − | + | ||
| + | === <span style=color:green>Word Problems</span> === | ||
| + | |||
| + | | | ||
|- | |- | ||
| | | | ||
| − | + | ==== <span style=color:green>"How Many" Problem</span> ==== | |
| + | |||
| + | | | ||
|- | |- | ||
| | | | ||
| − | + | *{{#annot:group of people|649|HhMp}}Question: a man passed by a group of people. | |
| − | | | + | :He said to them: hello one hundred people. |
| − | + | :They answered him: we are not one hundred people, but all of us, and other like us, and half of us, and a quarter of us with you will make one hundred. | |
| − | : | + | :<math>\scriptstyle X+X+\frac{1}{2}X+\frac{1}{4}X+1=100</math> |
| − | + | |style="text-align:right;"|<big>שאלה</big> אדם עבר על <s>אמר</s> אנשים אמר להם שלו' לכם מאה איש ענו אין אנו מאה איש אך אנו ואחרים כמונו ומחציתינו ורביעיתינו ועמך נהיה מאה{{#annotend:HhMp}} | |
| − | |||
| − | |||
| − | :<math>\scriptstyle | ||
| − | |style="text-align:right;"| | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::We take one for them; add one to them; add its half to them; it is two and a half. We add its quarter; they are 3-quarter. Since they are quarters added to integers, we convert the integers into quarters; [2] and 3-quarters are 11. |
| + | ::<math>\scriptstyle{\color{blue}{1+1+\frac{1}{2}+\frac{1}{4}=2+\frac{1}{2}+\frac{1}{4}=2+\frac{3}{4}=\frac{11}{4}}}</math> | ||
| + | |style="width:45%;text-align:right;"|נקח להם אחד נוסיף להם עוד אחד הנה נוסיף להם מחציתו הנה שנים וחצי נוסיף רביעיתו יהיה ג רביעי' ובעבור שהם רביעיות נוספות על השלמי' נשים גם השלמי' לרביעיות ויהיו ה וג' רביעיות הנה י"א | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Since he said that they are [100], their number without the addition of the one who spoke with them is 99. |
| + | |style="text-align:right;"|ובעבו' כי אמר שיהיו יהיה מספרם עם התוספת ט"ט לבד אותו שדבר עמהם | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::We convert the 99 into quarters; they are 396. |
| + | ::<math>\scriptstyle{\color{blue}{100-1=99=\frac{396}{4}}}</math> | ||
| + | |style="text-align:right;"|נחלק הט"ט שברים לרביעי' ויהיו שצ"ו | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::We divide them by 11; it is 36 and this is their number. |
| + | ::<math>\scriptstyle{\color{blue}{\frac{396}{4}\div\frac{11}{4}=\frac{396}{11}=36}}</math> | ||
| + | |style="text-align:right;"|נחלקם על י"א יהיו ל"ו וככה מספרם | ||
|- | |- | ||
| | | | ||
| − | + | ==== <span style=color:green>"If You Give Me" Problem</span> ==== | |
| − | + | ||
| | | | ||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *{{#annot:four men, item|664|xCtu}}Question: four buy one item. |
| + | :One said to his friends: each one will give half of what is in his purse and I will give everything I have in my purse and I will buy the item. | ||
| + | :The second [said]: each one will give a third of what is in his purse and I [will give] everything I have in my purse and I will buy the item. | ||
| + | :The third said: each one will give a quarter and I [will give] everything I have in my purse and I will buy the item. | ||
| + | :The fourth said: each one will give a fifth and I [will give] everything I have in my purse and I will buy the item. | ||
| + | :How much is the price of the item and how much does each have in his purse? | ||
| + | |style="text-align:right;"|<big>שאלה</big> ארבעה קונים חפץ אחד<br> | ||
| + | אחד אמר לחבירו כל אחד יתן החצי אשר בכיסו ואני אתן כל מה שיש בכיסי ואקנה החפץ<br> | ||
| + | והשיני כל אחד יתן שליש כל מה שבכיסו ואני כל מה בכיסי ואקנה החפץ<br> | ||
| + | והשלישי אמר כל אחד יתן הרביע ואני כל מה שבכיסי ואקנה החפץ<br> | ||
| + | והרביעי אמר כל אחד יתן חומש ואני כל מה שבכיסי ואקנה החפץ<br> | ||
| + | כמה ערך החפץ וכמה לכל אחד בכיסו{{#annotend:xCtu}} | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::The price of the item is 46 dinar. |
| + | |style="text-align:right;"|ערך החפץ מ"ו דינרי‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::The first has 15 pešiṭim. |
| + | |style="text-align:right;"|לראשון ט"ו פשוטי‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::The second has 23 dinar and 9 pešiṭim. |
| + | |style="text-align:right;"|ולשיני כ"ג דינרי' וט' פשיטי‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::The third has 31 dinar and 3 pešiṭim. |
| + | |style="text-align:right;"|לשלישי ל"א דינרי' ג פשיטי‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::The fourth has 35 dinar. |
| + | |style="text-align:right;"|לרביעי ל"ה דינרי‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::It is also possible to reach a half, a third and a quarter: the item 17 pešiṭim; the first - 5; the second - 11; and the third 13. |
| + | |style="text-align:right;"|ע"א להגיע עד חצי שליש ורביע החפץ י"ז פשיטי' לאחד ה' לשנים י"א לשלישי י"ג | ||
|- | |- | ||
| | | | ||
| − | + | ==== <span style=color:green>"Find the Amount" Problem</span> ==== | |
| − | + | ||
| | | | ||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *{{#annot:measures|630|w6l5}}Question: A man sells 13 measures for 23 pešiṭim. |
| + | :How many measures will he sell for 7 pešiṭim? | ||
| + | |style="text-align:right;"|<big>שאלה</big> אדם מכר י"ג מדות בכ"ג פשי‫'<br> | ||
| + | כמה מדות יתן בז' פש‫'{{#annotend:w6l5}} | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|' | + | ::As the ratio of 7 to 23 so is the ratio of the unknown to 13. |
| + | ::<math>\scriptstyle{\color{blue}{7:23=X:13}}</math> | ||
| + | |style="text-align:right;"|ערך ז' אל כ"ג כן ערך הנעלם אל י"ג | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::We multiply the extremes that are known; they are 91. |
| + | |style="text-align:right;"|נכפול הקצוות שהם נודעים יהיו צ"א | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::We divide them by 23; the result is 3 measures and 22 parts of 23 of one measure. |
| + | ::<math>\scriptstyle{\color{blue}{\frac{7\sdot13}{23}=\frac{91}{23}=3+\frac{22}{23}}}</math> | ||
| + | |style="text-align:right;"|נחלקם על כ"ג יעלו ג' מדות וכ"ב חלקי' מכ"ג במדה | ||
|- | |- | ||
| + | !<span style=color:green>"Find the Price" Problem</span> | ||
| | | | ||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *We reverse the matter: we want to know for how much will he sell 7 measures? |
| + | |style="text-align:right;"|ועוד נהפך הענין שנרצה לדעת בכמה יתן לו ז' מדות | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::We do like this: we multiply the extremes; they are 161. |
| + | |style="text-align:right;"|והנה נעשה הדמיון הזה נכפול הקצוות יהיו קס"א | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::We divide them by 1[3]; they are 12 pešiṭim and 5 parts of 13 of one pašiṭ. |
| + | ::<math>\scriptstyle{\color{blue}{\frac{7\sdot23}{13}=\frac{161}{13}=12+\frac{5}{13}}}</math> | ||
| + | |style="text-align:right;"|נחלקם על י"א והיו י"ב פשיטי' וה' חלקי' מי"ג בפשיט | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::If it is asked for how much will he sell 7 measures, the ratio of the unknown to 23 is the same as the ratio of 7 to 13. |
| + | ::<math>\scriptstyle{\color{blue}{X:23=7:13}}</math> | ||
| + | |style="text-align:right;"|אם שאל בכמה יתן ז' מדות יהיה ערך הנעלם בכ"ג אל כ"ג כערך ז' אל י"ג | ||
|- | |- | ||
| | | | ||
| − | + | ==== <span style=color:green>Pursuit Problem</span> ==== | |
| − | + | ||
| | | | ||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *{{#annot:two men|657|W30R}}Question: A man walks 29 miles a day. |
| + | :After 10 days, another starts walking 37 miles a day. | ||
| + | :When will he catch up with him? | ||
| + | |style="text-align:right;"|<big>שאלה</big> אדם הולך בכל יום כ"ט מילין<br> | ||
| + | אחר י' ימים נסע השני ההולך בכל יום ל"ז מילין<br> | ||
| + | מתי ישיגהו{{#annotend:W30R}} | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Multiply the miles he walks by 10 days; they are 290. |
| + | |style="text-align:right;"|כפול המילין שהולך בי' ימים יהיו ר"צ | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::We divide it by the difference between the two velocities, which is 8; it is 36 days and a quarter of a day. |
| + | ::<math>\scriptstyle{\color{blue}{\frac{29\sdot10}{8}=\frac{290}{8}=36+\frac{1}{4}}}</math> | ||
| + | |style="text-align:right;"|נחלקם על היתרון שיש בין שני המהלכים שהוא ח' והנה ל"ו ימים ורבע יום | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::As the ratio of 10 days to the unknown so is the ratio of the difference, which is 8, to 29. |
| + | ::<math>\scriptstyle{\color{blue}{10:X=8:29}}</math> | ||
| + | |style="text-align:right;"|הנה כערך י' ימים אל הנעלם כן ערך היתרון שהוא ח' אל כ"ט | ||
|- | |- | ||
| | | | ||
| − | + | ==== <span style=color:green>Encounter Problem</span> ==== | |
| + | |||
| + | | | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|' | + | *{{#annot:two walkers|658|DlW9}}Question: In how many days a 100 [miles] walk will be completed by two walkers at a [speed of] 19 and 17 towards each other? |
| + | |style="text-align:right;"|<big>שאלה</big> בכמה ימים יכלה מהלך ק' על ידי שני מהלכים של י"ט ושל י"ז זה לקראת זה{{#annotend:DlW9}} | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | ::It is easy to know that it is as the ratio of the sum of both to 100. Divide it by it; it is 2 and 28 [parts] of 36 [...]; 28 of 36 are 7-ninths of a day. |
| + | ::<math>\scriptstyle{\color{blue}{\frac{100}{19+17}=2+\frac{28}{36}=2+\frac{7}{9}}}</math> | ||
| + | |style="text-align:right;"|וקל לדעת כי הוא כערך מחובר שניהם אל קו וחלקם עליו יהיה ב' וכ"ח מל"ו כמו כן בב' ימים וכ"ח מל"ו ביום יכל ישהו לכלות וכ"ח מל"ו הם ז' תשיעיות היום | ||
|- | |- | ||
| − | | | + | | |
| − | + | ::If you want to know how many hours they are: it is known that the hours of the day are 12. | |
| − | |style="text-align:right;"|כי | + | |style="text-align:right;"|ואם תרצה לדעת כמה שעות הם ידוע הוא כי שעות היום י"ב |
|- | |- | ||
| − | | | + | | |
| − | :<math>\scriptstyle{\color{blue}{ | + | ::[As the ratio of] 7 to 9 so is the ratio of the unknown to 19. |
| − | + | ::<math>\scriptstyle{\color{blue}{7:9=X:19}}</math> | |
| − | + | |style="text-align:right;"|והנה ז' אל ט' כן ערך הנעלם אל י"ט | |
| − | |||
| − | : | ||
| − | |style="text-align:right;"| | ||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | + | ==== <span style=color:green>Payment Problem</span> ==== | |
| − | + | ||
| | | | ||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *{{#annot:three workers|612|NjKL}}Question: A man had 3 [workers]. |
| + | :[He said] that he would give the first 5 zehuvim and he would work for him for 20 days, but he did not want to finish the whole work. | ||
| + | :He said to the second that he would give him 4, but he did not want to finish [the work]. | ||
| + | :He said to the third that he would give him 5, but he did not want to finish either. | ||
| + | :The three of them went and did everything together and the employer paid each one equally. | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle\frac{5}{20}X=\frac{4}{20}Y=\frac{3}{20}Z\\\scriptstyle X+Y+Z=20\end{cases}</math> | ||
| + | |style="text-align:right;"|<big>שאלה</big> אדם עם ג' שיתן לראשון ה' זהובים ויעבוד לו כ' ימי' ולא רצה לגמור כל העבודה<br> | ||
| + | ואמר לשיני שיתן לו ד' ולא רצה לגמור<br> | ||
| + | אמר לשלישי שיתן לו ה' ולא רצה ג"כ לגמור אמר לשלישי שיתן לו ה' ולא רצה<br> | ||
| + | והלכו שלשתן ועשו הכל ביניהם והשוכר נתן לאחד אחד שוה{{#annotend:NjKL}} | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :This is similar to the question about the moneychanger, but we define the zehuvim divided among the workers as the coins, the hired work is the zahuv, the common denominator is 60 and the sum of the parts is 47. |
| + | |style="text-align:right;"|הנה זה דומה לשאלת המחליף אך נשים הזהובים המחולקי' לפועלי' במטבעות והעבודה הנשכרת היא הזהוב והמורה הוא ס' ומחובר החלקי' מ"ז | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|' | + | ::As the ratio of 47 to 60 so is the ratio of the zahuv to the unknown. |
| + | ::<math>\scriptstyle{\color{blue}{47:60=1:X}}</math> | ||
| + | |style="text-align:right;"|והנה כערך מ"ז אל ס' כן ערך הזהוב אל הנעלם | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::The part is worth one zahuv and 13 [parts] of 47 of one zahuv. |
| + | ::<math>\scriptstyle{\color{blue}{X=1+\frac{13}{47}}}</math> | ||
| + | |style="text-align:right;"|והנה החלק השוה זהו' אחד וי"ג ממ"ז בזהוב אחד | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::We wish to know: how much is the share of each: |
| + | |style="text-align:right;"|נבקש ערך כל אחד ב‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::The owner of the 3: 6 days and 2-thirds of a day [for one zahuv]. |
| + | |style="text-align:right;"|והנה בעל הג' הם ו' ימי' וב' שלישיות היום | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::The owner of the 4: 5 days for one zahuv. |
| + | |style="text-align:right;"|ובעל הד' בזהו' אחד ה' ימים | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::The owner of the 5: 4 days for one zahuv. |
| + | |style="text-align:right;"|ובעל הה' בזהוב אחד ד' ימי‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::The 3: divide his share by 47. As the ratio of 13 to 47 so is the ratio of the unknown to 20, which is the number of hours he works for one zahuv. |
| + | ::<math>\scriptstyle{\color{blue}{13:47=X:20}}</math> | ||
| + | |style="text-align:right;"|והנה של ג' עובד בשביל ח חלק העולה לו במ"ז כערך י"ג אל מ"ז כן ערך הנעלם אל כ' שהוא מספר שעות עבודתו בזהו' אחד | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::The 4: as the ratio of 13 to 47 so is the ratio of the unknown to [15]. |
| + | ::<math>\scriptstyle{\color{blue}{13:47=X:{\color{red}{15}}}}</math> | ||
| + | |style="text-align:right;"|ושל ד' כערך י"ג אל מ"ז כן ערך הנעלם אל מ"ח | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::If you add up all, the resulting sum is 20 days. |
| + | |style="text-align:right;"|ואם תחבר כל העולה בזה יעלה המחובר כ ימים | ||
|- | |- | ||
| | | | ||
| − | + | ||
| + | ==== <span style=color:Green>Boiling Problems</span> ==== | ||
| + | |||
| + | | | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|'' | + | :{{#annot:WP|663|D5pi}}Question: A man wanted to cook ten measures of must so that one-third remains. After 2 [measures] were reduced and 8 remained, 2 [measures] overflowed, so they were 6. Thus, one asks: how much should remain, so that it will be cooked according to the first plan, when it is known that at the beginning 3 and one-third should have been reduced? |
| + | :<math>\scriptstyle6:8=X:\left(3+\frac{1}{3}\right)</math> | ||
| + | |style="text-align:right;"|<big>שאלה</big> אדם אמר לבשל עשרה מדות מתירוש עד השאיר שליש לאחר שנתמעטו ב והיו ח נשפך ב והיו ו ושאל השואל כמה יהיה ראוי לישאר מן העומד לכ' לפנינו אם יבא לבשלו עד שיהיה כמשפט הראשון לפי מה שהתחיל כי ידוע שנתמעטו מג' ושליש{{#annotend:D5pi}} | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::As the ratio of 6 to 8 so is the ratio of the unknown to 3 and a third. |
| + | ::<math>\scriptstyle{\color{blue}{10:8=X:\left(3+\frac{1}{3}\right)}}</math> | ||
| + | |style="text-align:right;"|והנה כערך ו' אל ח' כן ערך הנעלם אל ג' ושליש | ||
|- | |- | ||
| | | | ||
| − | + | ==== <span style=color:Green>Whole from Parts Problems - lance</span> ==== | |
| + | |||
| + | | | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *{{#annot:lance|650|8OUv}}Question: a lance, a third of it is in the water, a quarter of it is in the soil, and [up above the water] it is 7 cubits [long]. |
| + | :What is the length of the whole [lance]? | ||
| + | :<math>\scriptstyle\frac{1}{3}X+\frac{1}{4}X+7=X</math> | ||
| + | |style="text-align:right;"|<big>שאלה</big> רומח מחציתו שלישיתו במים רביעיתו בעפר וגלוי ז אמות<br> | ||
| + | כמה גבהות כלו{{#annotend:8OUv}} | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::The common denominator is 12. |
| + | |style="text-align:right;"|הנה המורה י"ב | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::We subtract its parts from it; [the remainder] is 5. |
| + | ::<math>\scriptstyle{\color{blue}{12-\left(\frac{1}{3}\sdot12\right)-\left(\frac{1}{4}\sdot12\right)=12-7=5}}</math> | ||
| + | |style="text-align:right;"|נכסה ממנו חלקיו אלה יהיה הגלוי ה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::As the ratio of 5 to 12 so is the ratio of 7 to the unknown. |
| + | ::<math>\scriptstyle{\color{blue}{5:12=7:X}}</math> | ||
| + | |style="text-align:right;"|והנה כערך ה' אל י"ב כן ערך ז' אל הנעלם | ||
|- | |- | ||
| | | | ||
| − | + | ||
| − | + | ==== <span style=color:Green>Multiple Quantities Problems</span> ==== | |
| + | |||
| | | | ||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *{{#annot:men, women, children|652|R01b}}Question: A man ordered to give 20 pešuṭim to 20 people - men and women and children - he gave 2 pešiṭim to each man, a half to each woman, and [a quarter to each child]. |
| + | :How many [men, women, and children were there]? | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle2X+\frac{1}{2}Y+\frac{1}{4}Z=20\\\scriptstyle X+Y+Z=20\end{cases}</math> | ||
| + | |style="text-align:right;"|<big>שאלה</big> אדם צוה לתת כ' פשוט לכ' בני אדם אנשים ונשים ובנים לאיש נתן ב' פשיט ולאישה מחצה ולבנים [...]<br> | ||
| + | כמה לאיש{{#annotend:R01b}} | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::For 8 men - 16 pešiṭim. |
| + | |style="text-align:right;"|לח' אנשי' יו פשיטי‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::For 8 children - 2 pešiṭim |
| + | |style="text-align:right;"|לח' בני' ב' פשיטי‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::For 4 women - 2 pešiṭim. |
| + | |style="text-align:right;"|לד' נשי' ב' פשי‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|''' | + | *{{#annot:geese, pheasants, pullets|652|aHB5}}Question: A man said to his slave: Take 30 pešiṭim and buy me 30 poultry - a goose for 2 pešiṭim, a pheasant for a half, and a pullet for one pašiṭ. |
| + | :How many were there of each kind? | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle2X+\frac{1}{2}Y+Z=20\\\scriptstyle X+Y+Z=30\end{cases}</math> | ||
| + | |style="text-align:right;"|<big>שאלה</big> אדם אמר לעבדו קח ל' פשיטי' וקנה לי ל' עופות אווז ב' פשיטי' שליו מחצה פרגיות פשיט<br> | ||
| + | כמה יהיו מכל מין{{#annotend:aHB5}} | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::6 pullets, 8 geese, and 16 pheasants. |
| + | |style="text-align:right;"|ו' פרגיות ח' אווזות י"ו שלוים | ||
|- | |- | ||
| | | | ||
| − | + | ||
| + | ==== <span style=color:Green>Whole from Parts Problems - fish</span> ==== | ||
| + | |||
| + | | | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *{{#annot:fish|650|mZD5}}Question: a fish is 15 fingerbreadth [etzba'ot] in its body, without its tail and its head; its head is one-third of the whole fish and its tail is its quarter. How much is its whole with the head and the tail? |
| + | :<math>\scriptstyle15+\frac{1}{3}X+\frac{1}{4}X=X</math> | ||
| + | |style="text-align:right;"|<big>שאלה</big> דג יש לו טו אצבעות בגודל גופו לבד ראשו וזנבו וראשו מחזיק שלישית כל הדג והזנב מחזיק רביעיתו כמה מחזיק כלו עם הראש והזנב{{#annotend:mZD5}} | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :36 fingers: its third is 12; its quarter is 9. |
| + | |style="text-align:right;"|ל"ו אצבעות שלישיתו י"ב והרביעית ט | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :[?] you find that it is so. |
| + | |style="text-align:right;"|כפל החשבון שלישי' ורביעי' ותמצא כך | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *Question: A thief walks a fixed distance and the pursuer [walks] on the first day 1, on the second day 2 and so on he adds 2 every day, so the distance increases twice [the distance of] the thief. |
| + | :In how many days will he catch the thief walking the entire distance he walked each day? | ||
| + | |style="text-align:right;"|<big>שאלה</big> גנב הולך סכום אחד והרודף יום ראשון א ויום שיני ב וכן ויוסיף כל הימי' ב' כאשר יגדלו הסכום כפל הגנב ובתוך כך תפל אחד ובתוך כך סכום ימים ישיג הגנב בכל סכום שילך כמה בכל יום | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *Question: Shimon earned a seventh of his money on one day, the next day he earned a fifth of the whole amount, on the third day a third of the whole amount and the total sum is 10 dinar. |
| + | :We ask: how much is the amount of money? | ||
| + | :<math>\scriptstyle\frac{1}{7}X+\frac{1}{5}X+\frac{1}{3}X=10</math> | ||
| + | |style="text-align:right;"|<big>שאלה</big> שמעון הרויח שביעית ממנו היום ולמחר הרויח מן הכל חמישית ויום שלישי מן הכל שלישית והתחבר י' דינרי‫'<br> | ||
| + | ונשאל כמה הממון | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::The common denominator is 105. |
| − | + | |style="text-align:right;"|המורה ק"ה | |
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *Question: to weigh 60 with 11 stones; also [to pay] the rent for 4 years and 2 months with 18 rings |
| + | |style="text-align:right;"|<big>שאלה</big> לשקול ביא אבני' ס' [..] גם בי"ח טבעות לשכירות לד שנים וב חדשי‫' | ||
|- | |- | ||
| | | | ||
| − | + | ::The result for the weight is with doubles. | |
| − | + | |style="text-align:right;"|יצא בכפל למשקל | |
| − | |||
| − | |||
| − | : | ||
| − | |||
| − | |style="text-align:right;"| | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Do for rings as follows: 1, 2, 4, 8, 16. |
| − | + | |style="text-align:right;"|וכן תעשה בטבעות א ב ד ח י"ו | |
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Duplicate until the number is obtained. |
| − | + | |style="text-align:right;"|וכפול עד שיעלה החשבון | |
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::For stones the result is with triples: 1, 3, 9, 27 |
| − | + | |style="text-align:right;"|ובאבנים יצא בשלישות א ג ט כז | |
|- | |- | ||
| | | | ||
| − | + | ||
| − | + | == Glosses on Abraham Ibn Ezra’s Book of the number (P1026) == | |
| − | |||
| − | |||
| | | | ||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | === The author of the Sefer Yezira === |
| + | |||
| + | |style="text-align:right;"|[[ספר_המספר_/_אברהם_אבן_עזרא#author of the Sefer Yezira|<span style=color:blue>'''ובעל ספר יצירה'''</span>]] | ||
|- | |- | ||
| − | | | + | |Explanation: The intention of the author of Sefer Yezira [= the Book of Creation] is to inform how man was created by one act from the creatures. |
| − | + | |style="text-align:right;"|פי' בעל כוונת ספר יצירה להודיע איך נברא האדם בתחבולה אחת מין הנבראים | |
| − | |style="text-align:right;"|' | ||
| − | |||
|- | |- | ||
| − | | | + | |He said that three matters were needed for this: |
| − | |style="text-align:right;"|''' | + | |style="text-align:right;"|ואמ' שיצטרכו בו ג' עניני‫' |
|- | |- | ||
| | | | ||
| − | : | + | |
| − | |style="text-align:right;"|''' | + | === Sefar === |
| + | |||
| + | The writing: through the faculties of the letters that are indicating him, the creation is done by the letters. | ||
| + | |style="text-align:right;"|[[ספר_המספר_/_אברהם_אבן_עזרא#Sefar|<span style=color:blue>'''הספר'''</span>]] הוא הכתיבה כי בכחות האותיות יורו בו באותיות יעשה היצירה | ||
|- | |- | ||
| | | | ||
| − | + | ||
| − | |style="text-align:right;"| | + | === Sefer === |
| + | |||
| + | It is the number, because composition and merging are only done by that one number is the duplication of the other. | ||
| + | |style="text-align:right;"|[[ספר_המספר_/_אברהם_אבן_עזרא#Sefer|<span style=color:blue>'''וספר'''</span>]] הוא המנין כי ההרכבה והמזגה לא תעשה רק במספר שבאחד מהם יהיה בו מיסוד האחד כפל האחר | ||
|- | |- | ||
| | | | ||
| − | + | ||
| − | + | === Sipur === | |
| − | |style="text-align:right;"|כי | + | |
| + | Because the scholars of this science assumed that they were leading down a spiritual force that gave power to that composition, so that the action of this power is not through anything, but not through anything is possible only through God. | ||
| + | |style="text-align:right;"|[[ספר_המספר_/_אברהם_אבן_עזרא#Sipur|<span style=color:blue>'''וספור'''</span>]] כי חכמי חכמה זו הניחו שהם יורידו כח אשר נתן כח בהרכבה ההיא עד שיעשה פעל הכח בלתי מדבר אבל לא מדבר כי זה לא יתכן אלא לאל ית‫' | ||
|- | |- | ||
| | | | ||
| − | :: | + | |
| − | : | + | === Since === |
| − | |style="text-align:right;"| | + | |
| + | |style="text-align:right;"|[[ספר_המספר_/_אברהם_אבן_עזרא#Since God|<span style=color:blue>'''בעבור'''</span>]] | ||
| + | |- | ||
| + | |The word "since" is applied until his saying below, "Therefore, the scales of every number". | ||
| + | |style="text-align:right;"|מלת בעבור נמשכת עד אמרו למטה על כן כל מאזני כל מספר | ||
| + | |- | ||
| + | |Sefar is the composite. | ||
| + | |style="text-align:right;"|'''ספר''' הוא המרוכב | ||
| + | |- | ||
| + | |Sefer is the number, as "after the numbering wherewith [David his father] had numbered them [Chronicles 2, 2, 16]. | ||
| + | |style="text-align:right;"|'''וספר''' הוא המספר כמו ''אחר הספר אשר ספרם''‫<ref group=note>דברי הימים ב, ב, טז</ref> | ||
|- | |- | ||
| − | | | + | |Sipur is the expression of units. |
| − | + | |style="text-align:right;"|'''וספור''' הוא הדבור באחדים | |
| − | |||
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | ::We subtract 6 from 48; 42 remains and this is the amount of money he has. | + | |
| − | ::<math>\scriptstyle{\color{blue}{48-6=42}}</math> | + | === Higher decade === |
| − | |style="text-align:right;"|נגרע מספר ו' ממ"ח וישאר מ"ב והם המעות שבידו | + | |
| − | |- | + | |style="text-align:right;"|[[ספר_המספר_/_אברהם_אבן_עזרא#higher decade|<span style=color:blue>'''בכלל הגבוה'''</span>]] |
| − | | | + | |- |
| − | :*Or: he spends 18 pešiṭim every day and at the end of five days he has nothing left. | + | |Meaning: the closest to it [above] it. |
| − | |style="text-align:right;"|או שיוציא בכל יום י"ח פשי' ולסוף ה' ימים לא נשאר לו מאומה | + | |style="text-align:right;"|פי' הקרוב לו לפניו |
| − | |- | + | |- |
| − | | | + | | |
| − | ::One half and one part of 16, which is 9 parts of 16, is the number that when doubled in a double ratio as many times as the number of days, which is 5, the result is 18. | + | |
| − | |style="text-align:right;"|הנה המספר שבהכפלו על יחס הכפל פעמים כמספר הימים שהוא ה' יעלה י"ח הוא חצי וחלק אחד מי"ו שהן ט' חלקים מי"ו | + | === The remainder is the required === |
| − | |- | + | |
| − | | | + | |style="text-align:right;"|[[ספר_המספר_/_אברהם_אבן_עזרא#the remainder is the required|<span style=color:blue>'''והנשאר הוא המבוקש'''</span>]] |
| − | ::Because, when we double one half and one part of 16, the result is 1 and one-eighth. | + | |- |
| − | ::<math>\scriptstyle{\color{blue}{2\sdot\left(\frac{1}{2}+\frac{1}{16}\right)=1+\frac{1}{8}}}</math> | + | |Explanation: a third multiplied by a third is a ninth. |
| − | |style="text-align:right;"|כי כשנכפול חצי וחלק אחד מי"ו יעלה א' ושמינית | + | :<math>\scriptstyle{\color{blue}{\frac{1}{3}\times\frac{1}{3}=\frac{1}{9}}}</math> |
| − | |- | + | |style="text-align:right;"|פי' שהשלישית הנכפל על השלישית יצא תשיעית |
| − | | | + | |- |
| − | ::When we double 1 and one-eighth, the result is 2 and a quarter. | + | |Therefore, when we multiply a third of a number by its third, we receive a ninth of the required square. |
| − | ::<math>\scriptstyle{\color{blue}{2\sdot\left(1+\frac{1}{8}\right)=2+\frac{1}{4}}}</math> | + | :<math>\scriptstyle{\color{blue}{\left(\frac{1}{3}\sdot a\right)\times\left(\frac{1}{3}\sdot a\right)=\frac{1}{9}a^2}}</math> |
| − | |style="text-align:right;"|וכשנכפול א' ושמינית יעלה ב' ורביע | + | |style="text-align:right;"|אם כן בכפלנו שליש המספר על שלישו יצא לנו תשיעית מרובע הדרוש |
| − | |- | + | |- |
| − | | | + | |When we take the same in the higher rank, we receive ten times the ninth of the square. |
| − | ::When we double 2 and a quarter, the result is 4 and a half. | + | |style="text-align:right;"|וכאשר לקחנו כמוהו בכלל הגבוה ממנו יצא לנו עשרה דמיוני תשיעית המרובע |
| − | ::<math>\scriptstyle{\color{blue}{2\sdot\left(2+\frac{1}{4}\right)=4+\frac{1}{2}}}</math> | + | |- |
| − | |style="text-align:right;"|וכשנכפול ב' ורביע יעלה ד' וחצי | + | |Therefore, when we subtract from it the square of the third, which is a ninth of the square, the remainder equals the required square. |
| − | |- | + | |style="text-align:right;"|אם כן כשחסרנו מזה מרובע השליש שהוא תשיעית המרובע ישאר שוה למרובע הדרוש |
| − | | | + | |- |
| − | ::When we double 4 and a half, the result is 9. | + | | colspan=2| |
| − | ::<math>\scriptstyle{\color{blue}{2\sdot\left(4+\frac{1}{2}\right)=9}}</math> | + | :<math>\scriptstyle{\color{blue}{10\sdot\left[\left(\frac{1}{3}\sdot a\right)\times\left(\frac{1}{3}\sdot a\right)\right]-\left[\left(\frac{1}{3}\sdot a\right)\times\left(\frac{1}{3}\sdot a\right)\right]=\left(10\sdot\frac{1}{9}a^2\right)-\frac{1}{9}a^2=a^2}}</math> |
| − | |style="text-align:right;"|וכשנכפול ד' וחצי יעלה ט‫' | + | |- |
| − | |- | + | | |
| − | | | + | |
| − | ::And when we double 9 for the fifth time, the result is 18. | + | === The sum is the required === |
| − | ::<math>\scriptstyle{\color{blue}{2\sdot9=18}}</math> | + | |
| − | |style="text-align:right;"|וכשנכפול חמשית ט' יעלה י"ח | + | |style="text-align:right;"|[[ספר_המספר_/_אברהם_אבן_עזרא#the sum is the required|<span style=color:blue>'''והמחובר הוא הדרוש'''</span>]] |
| − | |- | + | |- |
| + | | | ||
| + | *Explanation: although the author mentioned this method only for extracting squares, it can be applied to the product of any two numbers divisible by three. | ||
| + | |style="text-align:right;"|פי' אעפ"י שלא זכר המחבר דרך זו רק בהוצאת המרובעים יתכן זה בכפל כל שני מספרים בעלי שליש | ||
| + | |- | ||
| + | | | ||
| + | :*Such as 12 and 18. | ||
| + | ::<math>\scriptstyle{\color{blue}{12\times18}}</math> | ||
| + | |style="text-align:right;"|כמו י"ב וי"ח | ||
| + | |- | ||
| + | | | ||
| + | ::We multiply a third of the one, which is 4, by the third of the other, which is 6; the result is 24. | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(\frac{1}{3}\sdot12\right)\times\left(\frac{1}{3}\sdot18\right)=4\times6=24}}</math> | ||
| + | |style="text-align:right;"|אנו נכפול שליש האחד והוא ד' בשליש השני והוא ו' ויעלה כ"ד | ||
| + | |- | ||
| + | | | ||
| + | ::We rise it one rank higher; it is 240. | ||
| + | |style="text-align:right;"|נגביההו מעלה אחת ויהיה ר"מ | ||
| + | |- | ||
| + | | | ||
| + | ::Subtract the product of the two-thirds, which is 24; 216 remains and this is the product of 18 by 12. | ||
| + | |style="text-align:right;"|הסיר ‫<ref>76r</ref>כפל השני שלישיות שהוא כ"ד ישארו רי"ו והוא כפל י"ח על י"ב | ||
| + | |- | ||
| + | | colspan=2| | ||
| + | ::<math>\scriptstyle{\color{blue}{12\times18=10\sdot\left[\left(\frac{1}{3}\sdot12\right)\times\left(\frac{1}{3}\sdot18\right)\right]-\left[\left(\frac{1}{3}\sdot12\right)\times\left(\frac{1}{3}\sdot18\right)\right]=10\sdot\left(4\times6\right)-\left(4\times6\right)=240-24=216}}</math> | ||
| + | |- | ||
| + | | | ||
| + | *If they [are not divisible by three], but exceed by one: | ||
| + | |style="text-align:right;"|ואם שהם נעדרי השליש ומוסיפין אחד | ||
| + | |- | ||
| + | | | ||
| + | :*As 13 and 19, we do as follows: | ||
| + | ::<math>\scriptstyle{\color{blue}{13\times19}}</math> | ||
| + | |style="text-align:right;"|כמו י"ג י"ט נעש[ה] כן | ||
| + | |- | ||
| + | | | ||
| + | ::We calculate the product of 12 by 18; the result is 216. | ||
| + | ::<math>\scriptstyle{\color{blue}{12\times18=216}}</math> | ||
| + | |style="text-align:right;"|נוציא סך החשבון לי"ב וי"ח ויעלה רי"ו | ||
| + | |- | ||
| + | | | ||
| + | ::Since each of the numbers exceed by one over [the divisible by three], we add 12, 18, and one; the result is 247 and this is the product of 13 by 19. | ||
| + | |style="text-align:right;"|ולפי שכל אחד מהמספרים מוסיף אחד על השליש נוסיף י"ב וי"ח ואחד ועלה רמ"ז וזהו כפל י"ג בי"ט | ||
| + | |- | ||
| + | | colspan=2| | ||
| + | ::<math>\scriptstyle{\color{blue}{13\times19=\left(12\times18\right)+12+18+1=216+12+18+1=247}}</math> | ||
| + | |- | ||
| + | | | ||
| + | *If the two numbers are one less [than the divisible by three]: | ||
| + | |style="text-align:right;"|ואם היו שני המספרים חסרין אחד | ||
| + | |- | ||
| + | | | ||
| + | :*As 11 and 17: | ||
| + | ::<math>\scriptstyle{\color{blue}{11\times17}}</math> | ||
| + | |style="text-align:right;"|כי"א וי"ז | ||
| + | |- | ||
| + | | | ||
| + | ::We calculate the product of 12 by 18; the result is 216. | ||
| + | ::<math>\scriptstyle{\color{blue}{12\times18=216}}</math> | ||
| + | |style="text-align:right;"|נעשה החשבון בי"ב וי"ח ויעלה רי"ו | ||
| + | |- | ||
| + | | | ||
| + | ::We subtract 11, 17 and 1 from 216 for the deficiency; 187 remains and this is the product of 11 by 17. | ||
| + | |style="text-align:right;"|ובעד חסרון האחד נגרע י"א וי"ז ואחד מן רי"ו וישאר קפ"ז והוא כפל י"א בי"ז | ||
| + | |- | ||
| + | | colspan=2| | ||
| + | ::<math>\scriptstyle{\color{blue}{11\times17=\left(12\times18\right)-11-17-1=216-11-17-1=187}}</math> | ||
| + | |- | ||
| + | | | ||
| + | *If one exceeds by one and the other is less by one: | ||
| + | |style="text-align:right;"|ואם האחד מוסיף אחד והשני חוסר אחד | ||
| + | |- | ||
| + | | | ||
| + | ::As 11 and 19, or 13 and 17. | ||
| + | |style="text-align:right;"|כי"א וי"ט או י"ג וי"ז | ||
| + | |- | ||
| + | | | ||
| + | ::We calculate the product of 12 by 18; the result is 216. | ||
| + | ::<math>\scriptstyle{\color{blue}{12\times18=216}}</math> | ||
| + | |style="text-align:right;"|נעשה החשבון בי"ב וי"ח ויעלה רי"ו | ||
| + | |- | ||
| + | | | ||
| + | :*If they are 11 and 19: | ||
| + | ::<math>\scriptstyle{\color{blue}{11\times19}}</math> | ||
| + | |style="text-align:right;"|הנה אם היו י"א וי"ט | ||
| + | |- | ||
| + | | | ||
| + | ::The [deficient] is 11 and the [exceeding] is 1[9], so the [exceeding] is greater than the [deficient] by [8; minus 1; it is] 7. Subtract it from 216; 209 remains and this is the required. | ||
| + | |style="text-align:right;"|התוספת הוא י"א והחסרון הוא י"ח אם כן החסרון רב על התוספת ז' חסרם מן רי"ו וישאר ר"ט והוא הדרוש | ||
| + | |- | ||
| + | | colspan=2| | ||
| + | ::<math>\scriptstyle{\color{blue}{11\times19=\left(12\times18\right)-\left(19-11-1\right)=216-7=209}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :*If they are 13 and 17: | ||
| + | ::<math>\scriptstyle{\color{blue}{13\times17}}</math> | ||
| + | |style="text-align:right;"|ואם היו י"ג וי"ז | ||
| + | |- | ||
| + | | | ||
| + | ::The [deficient] is 17 and the [exceeding] is 13, so the excess of the greater is [4; plus 1; it is] 5. We add it to 216; it is 221 and this is the required. | ||
| + | |style="text-align:right;"|הנה התוספת הוא י"ז והחסרון י"ג אם כן התוספת רב ה' נוסיפם על רי"ו והיה רכ"א והוא הדרוש | ||
| + | |- | ||
| + | | colspan=2| | ||
| + | ::<math>\scriptstyle{\color{blue}{17\times13=\left(12\times18\right)+\left(17-13+1\right)=216+5=221}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :*If they are 18 and 13: | ||
| + | ::<math>\scriptstyle{\color{blue}{18\times13}}</math> | ||
| + | |style="text-align:right;"|ואם הן כמו י"ח וי"ג | ||
| + | |- | ||
| + | | | ||
| + | ::We add 18 to 216; it is 23[4] and this is the required. | ||
| + | |style="text-align:right;"|נוסיף על רי"ו י"ח והיה רל"ב והוא הדרוש | ||
| + | |- | ||
| + | | colspan=2| | ||
| + | ::<math>\scriptstyle{\color{blue}{18\times13=\left(12\times18\right)+18=216+18=234}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :*If they are 18 and 11: | ||
| + | ::<math>\scriptstyle{\color{blue}{18\times11}}</math> | ||
| + | |style="text-align:right;"|ואם הן י"ח וי"א | ||
| + | |- | ||
| + | | | ||
| + | ::We subtract 18 from 216; 198 remains. | ||
| + | |style="text-align:right;"|נגרע י"ח מן רי"ו וישאר קצ"ח | ||
| + | |- | ||
| + | | colspan=2| | ||
| + | ::<math>\scriptstyle{\color{blue}{18\times11=\left(12\times18\right)-18=216-18=198}}</math> | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | === Example: we wish to multiply 29 by 31 === | ||
| + | |||
| + | |style="text-align:right;"|[[ספר_המספר_/_אברהם_אבן_עזרא#29_by_31|<span style=color:blue>'''דמיון רצינו לכפול כ"ט על ל"א'''</span>]] | ||
| + | |- | ||
| + | | | ||
| + | :Explanation: another example: when we wish to multiply, for instance, 8 by 12, whose distance from 10, which is a decade, is the same, which is 2. | ||
| + | :<math>\scriptstyle{\color{blue}{8\times12}}</math> | ||
| + | |style="text-align:right;"|פי' דמיון אחר ברצותנו לכפול כמשל ח' על י"ב שמרחקם מי' שהוא כלל מספר שוה והוא ב‫' | ||
| + | |- | ||
| + | | | ||
| + | :We multiply 10 by itself; it is 100. | ||
| + | :<math>\scriptstyle{\color{blue}{10\times10=100}}</math> | ||
| + | |style="text-align:right;"|נכפול י' על עצמו והיה ק‫' | ||
| + | |- | ||
| + | | | ||
| + | :We subtract 4 from it, which is the square of 2. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(10\times10\right)-\left(2\times2\right)=100-4}}</math> | ||
| + | |style="text-align:right;"|נחסר ממנו ד' שהוא מרובע ב‫' | ||
| + | |- | ||
| + | | | ||
| + | :The explanation of this is that the multiplication of 8 by 12 is the multiplication of 8 by 10 and by 2. | ||
| + | :<math>\scriptstyle{\color{blue}{8\times12=\left(8\times10\right)+\left(8\times2\right)}}</math> | ||
| + | |style="text-align:right;"|ובאור זה שכפל ח' על י"ב הוא כפל ח' על י' ועל ב‫' | ||
| + | |- | ||
| + | | | ||
| + | :The multiplication of 10 by 10 consists of the multiplication of 10 by 8 and by 2. | ||
| + | :<math>\scriptstyle{\color{blue}{10\times10=\left(10\times8\right)+\left(10\times2\right)}}</math> | ||
| + | |style="text-align:right;"|וכפל י' על י' הוא כולל כפל י' על ח' ועל ב‫' | ||
| + | |- | ||
| + | | | ||
| + | :The multiplication of 10 by 2 consists of the multiplication of 8 by 2 and 2 by 2. | ||
| + | :<math>\scriptstyle{\color{blue}{10\times2=\left(8\times2\right)+\left(2\times2\right)}}</math> | ||
| + | |style="text-align:right;"|וכפל י' על ב' הוא כולל כפל ח' על ב' וב' על ב‫' | ||
| + | |- | ||
| + | | | ||
| + | :We find that the excess of 10 by 10 over 8 by 12 is 2 by 2. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(10\times10\right)-\left(8\times12\right)=2\times2}}</math> | ||
| + | |style="text-align:right;"|נמצא יתרון י' על י' מן ח' על י"ב הוא ב' על ב‫' | ||
| + | |- | ||
| + | |From this you understand all the other examples, and this is according to proposition 6 of Euclid's Book II. | ||
| + | |style="text-align:right;"|ומזה תבין לכל המשלים האחרים וזה מתמונת ו' ממאמר ב' לאיקלידיס | ||
| + | |- | ||
| + | |The rule is the same for any two different numbers, even if there are no decades: we add to the smaller number half the difference between the two numbers, square [the sum], subtract from the square the square of half the difference and the remainder is equal to the product of one of the two numbers by the other. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{a\times b=\left[a+\frac{1}{2}\sdot\left(b-a\right)\right]^2-\left[\frac{1}{2}\left(b-a\right)\right]^2}}</math> | ||
| + | |style="text-align:right;"|וכן ההנהגה בכל שני מספרים מתחלפים אך כי לא יהיו כללים שנוסיף על המספר היותר קטן חצי יתרון שבין שני המספרים ונרבעהו ונחסר מהמרובע מרובע חצי היתרון והנשאר ישוה להכאת אחד משני המספרים ‫<ref>76v</ref>באחר | ||
| + | |- | ||
| + | |But this scholar wanted to make it easier, not harder, and for this reason he explained it only for the numbers whose distance from a decade is the same. | ||
| + | |style="text-align:right;"|אך זה החכם בא להקל ולא להחמיר ולזה לא באר במספרים שמרחקן מחשבון כלל מספר שוה | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | === Know that if there are two digits to multiply === | ||
| + | |||
| + | |style="text-align:right;"|[[ספר_המספר_/_אברהם_אבן_עזרא#one digit by one digit|<span style=color:blue>'''ודע כי אם יהיו שני מספרים לכפול'''</span>]] | ||
| + | |- | ||
| + | |Explanation: if you wish to multiply 2 by 40, it is just a multiplication of one rank by another, but if we have one digit by two digits, or two by two, the ranks must be multiplied by each other according to the numerical value of the digits. | ||
| + | |style="text-align:right;"|פי' כי אם תרצה להכות ב' על מ' אין כאן הכאה רק ממדרגה האחת על האחרת אך אם יהיה לנו מספר על שני מספרים או שנים על שנים אז צריך לכפול המעלות זו על זו כפי מנין המספרים | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | === You have to === | ||
| + | |||
| + | |style="text-align:right;"|[[ספר_המספר_/_אברהם_אבן_עזרא#two digits by two digits|<span style=color:blue>'''אתה צריך'''</span>]] | ||
| + | |- | ||
| + | |Explanation: he said it in actu, although sometimes it can be done with less, because he said above: if they are two by two, it should be done 4 times, and then he explained that it can be done with less, so here too it can be done with less. Understand. | ||
| + | |style="text-align:right;"|פי' אמ' זה בשלוח אעפי שלפעמים יתכן בפחות מזה כי כן אמר למעלה ואם הם שנים על שנים צריך לעשות זה ד' פעמים ואחר כן באר שאפשר בפחות וגם בזה אפשר בפחות ובין | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | === As if they are units === | ||
| + | |||
| + | |style="text-align:right;"|[[ספר_המספר_/_אברהם_אבן_עזרא#as if they are units|<span style=color:blue>'''כאלו הם אחדים'''</span>]] | ||
| + | |- | ||
| + | |Explanation: the reason for this is that every decade exceeds by one over the ninths, therefore we have to consider each decade as one. | ||
| + | |style="text-align:right;"|פי' וסבת זה כי כל כלל יוסיף אחד על תשיעיות וכן שנים <s>על</s> שנים וכן כלן אם כן לנו שנחשב אחד לכל כלל | ||
| + | |- | ||
| + | |The reason for this is that the foundation of number are 9 numbers and here their units are inclusive. This is his statement at the beginning of the book: "therefore the scales of the number are 9". | ||
| + | |style="text-align:right;"|וסבת זה היות ט' מספרים יסוד החשבון והכא אחדיהם הוא כולל והוא אמרו בראש הספר על כן היו מאזני המספר ט‫' | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | === Does not assume any change === | ||
| + | |||
| + | |style="text-align:right;"|[[ספר_המספר_/_אברהם_אבן_עזרא#one_does_not_assume_change|<span style=color:blue>'''לא יקבל שנוי'''</span>]] | ||
| + | |- | ||
| + | |Explanation: because change comes from composition, and the one is simple from being one. | ||
| + | |style="text-align:right;"|פי' כי השנוי יבא מצד ההרכבה והאחד פשוט כי מהיותו אחד | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | === Does not assume any increase === | ||
| + | |||
| + | |style="text-align:right;"|[[ספר_המספר_/_אברהם_אבן_עזרא#one_does_not_assume_increase|<span style=color:blue>'''לא יקבל רבוי'''</span>]] | ||
| + | |||
| + | |- | ||
| + | |Because 1 [multiplied] by 1 is 1. | ||
| + | |style="text-align:right;"|כי א' על א' הוא א‫' | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | === Does not assume any division === | ||
| + | |||
| + | |style="text-align:right;"|[[ספר_המספר_/_אברהם_אבן_עזרא#one_does_not_assume_division|<span style=color:blue>'''ולא חלוק'''</span>]] | ||
| + | |- | ||
| + | |Because, if you divide it, there are two. | ||
| + | |style="text-align:right;"|כי אם תחלקהו יהיו שנים | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | === It is the cause of any change === | ||
| + | |||
| + | |style="text-align:right;"|[[ספר_המספר_/_אברהם_אבן_עזרא#one_is_the_cause_of_increase_and_change|<span style=color:blue>'''והוא סבת כל שנוי'''</span>]] | ||
| + | |- | ||
| + | |Because the change results from the combination of two matters and the same for the number. | ||
| + | |style="text-align:right;"|כי בהרכבת שני ענינים יבא השנוי וכן במספר | ||
| + | |- | ||
| + | | | ||
| + | === According to its rank === | ||
| + | |||
| + | |style="text-align:right;"|[[ספר_המספר_/_אברהם_אבן_עזרא#each_according_to_its_rank|<span style=color:blue>'''כפי מעלתו'''</span>]] | ||
| + | |- | ||
| + | |Explanation: units corresponding to units and tens corresponding to tens. | ||
| + | |style="text-align:right;"|פי' אחדים כנגד אחדים ועשרות כנגד עשרות | ||
| + | |- | ||
| + | | | ||
| + | === Less than the number === | ||
| + | |||
| + | |style="text-align:right;"|[[ספר_המספר_/_אברהם_אבן_עזרא#the_divisor_should_be_less_than_the_dividend|<span style=color:blue>'''ופחות מהמספר'''</span>]] | ||
| + | |- | ||
| + | |Explanation: the dividend number should be greater than the divisor number, because it is like the measure and the measured. | ||
| + | |style="text-align:right;"|פי' שהמספר המחולק ראוי להיות יותר גדול מהמספר המחלק כי זה כמו המדה עם הנמדד | ||
| + | |- | ||
| + | |More precisely, this is not mandatory, as will be explained regarding the division of fractions, when we divide five-ninths by a whole number. | ||
| + | |style="text-align:right;"|וזה ביותר נכון לא מחויב כמו שיתבאר בחלוק השברים שנחלק חמש תשיעיות על שלם | ||
| + | |- | ||
| + | | | ||
| + | === Did not reach the rank of the units === | ||
| + | |||
| + | |style="text-align:right;"|[[ספר_המספר_/_אברהם_אבן_עזרא#not_reached_the_units|<span style=color:blue>'''ולא הגיע למעלת האחדים'''</span>]] | ||
| + | |- | ||
| + | |Explanation: the result of division has not yet reached the rank of the units, because, if it had, it would have already been completely gone and would no longer be subject to division, since the dividend has already been divided into a number smaller than the divisor. | ||
| + | |style="text-align:right;"|פי' שהיוצא בחלוק לא הגיע למעלת האחדים שאם היה כן כבר יצא לחוץ ולא יקבל עוד החלוקה שכבר נתך המחולק אל מספר יותר קטן מהמחולק עליו | ||
| + | |- | ||
| + | |By saying: not subject to division, i.e. for integers, but for fractions, it is subject to division. Understand. | ||
| + | |style="text-align:right;"|ובאמרנו לא יקבל החלוקה ר"ל בשלמים אך בשברים יקבל החלוק ובין | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | === Not gone completely === | ||
| + | |||
| + | |style="text-align:right;"|‫<ref>77r</ref>[[ספר_המספר_/_אברהם_אבן_עזרא#not_gone_completely|<span style=color:blue>'''כי לא יצא לחוץ'''</span>]] | ||
| + | |- | ||
| + | |Explanation: decomposing the dividend until it reaches a number smaller than the divisor, is called completely gone [lit. exiting]. | ||
| + | |style="text-align:right;"|פי' התכת המחולק עד שיגיע למספר יותר קטן מהמחולק עליו יקרא יציאה לחוץ | ||
| + | |- | ||
| + | |If it is divided [..], it reaches outside the rank of units, therefore it becomes fractions, ten of which is one whole, and they are called primes. Every prime is divided into 10 seconds. He did not aim only to the division of integers by integers. | ||
| + | |style="text-align:right;"|שאם נחלק [..]יצא לחוץ ממעלת האחדים ויהיו שברים אשר העשרה מהם הוא אחד שלם יקראו ראשוניים וכן כל ראשון יחלק לי' שניים והוא לא כיון רק בחלוק שלמים על שלמים | ||
| + | |- | ||
| + | | | ||
| + | === We always subtract backwards === | ||
| + | |||
| + | |style="text-align:right;"|[[ספר_המספר_/_אברהם_אבן_עזרא#we_always_subtract_backwards|<span style=color:blue>'''ולעולם נחזור אחורנית'''</span>]] | ||
| + | |- | ||
| + | |It is necessary to investigate what brought him to this, because it is more correct and easier to subtract directly. | ||
| + | |style="text-align:right;"|צ"ע מה הביאו לזה כי יותר נכון ונקל לחסר ביושר | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | === One is like a point === | ||
| + | |||
| + | |style="text-align:right;"|[[ספר_המספר_/_אברהם_אבן_עזרא#one_is_as_a_point_in_a_circle|<span style=color:blue>'''האחד כנקדה'''</span>]] | ||
| + | |- | ||
| + | |Explanation: because the point is like the foundation of the circle because the one who draws a circle, before drawing it, draws a center, on which he establishes the circumference of the circle. | ||
| + | |style="text-align:right;"|פי' כי הנקדה כיסוד לעגול כי המקוה לעגול טרם הקוותו יניח מוצק עליה יכונן רחב העגול | ||
| + | |- | ||
| + | |The one is the foundation of the number, and therefore it cannot be divided like the point, since it is one. | ||
| + | |style="text-align:right;"|וכן האחד הוא יסוד המספר ולכן לא יתכן לחלק כמו הנקדה מצד שהוא אחד | ||
| + | |- | ||
| + | |But since every sum has the name of one, being composed of many individuals, like the body, which is composed of four phlegms and other organs, which have homogeneous parts, and the hand and the arm, and the rest of the organs, hence it is undoubtedly possible for it to be decomposed into parts, except for the intellect, in which the speech is created, for this reason it was decided that the simple division is done in the intellect, because without the intellect it is impossible in any way. | ||
| + | |style="text-align:right;"|ואולם מצד ההוה כל כלל בשם אחד עם היותו מורכב מאישים רבים כמו הגוף המורכב מד' ליחות ומשאר אברים מתדמי החלקים ומהיד והזרוע ושאר אברים הכליים והנה הוא בלי ספק יתכן בו התפרקו לחלקו חוץ לשכל יתחדש בו הדבור בעבור זה החליטו החלוק הפשוט להעשותו בו בשכל כי חוץ לשכל אי אפשר בשום פנים | ||
| + | |- | ||
| + | |Another explanation: all this is an explanation for dividing the one into fractions, and just as the point is an indivisible thing and all lines are drawn from it, so is the one, and for this reason it cannot be divided. | ||
| + | |style="text-align:right;"|עוד פי' כל זה הצעה לחלוקת האחד לשברים וכמו שהנקדה היא דבר בלתי מתחלק וכל הקוים יוצאים ממנה כן האחד ולזה לא היה ראוי לחלק | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | === Consists of surfaces === | ||
| + | |||
| + | |style="text-align:right;"|[[ספר_המספר_/_אברהם_אבן_עזרא#the_body_consists_of_surfaces|<span style=color:blue>'''מורכב משטחים'''</span>]] | ||
| + | |- | ||
| + | |Explanation: he did not mean that the body is a chemical composition of surfaces and not of lines and points, as explained in the physics books, but in his statement he meant the surfaces of the encompassing objects and many in the human body, such as the skull that is encompassed by one surface, and so are many organs, and the body is called one. | ||
| + | |style="text-align:right;"|פי' אין כונתו שהגוף הרכבה מזגיית משטחים ולא מקוים ונקדות כמבואר בספרי הטבע אך כונתו באמרו שטחים התכליות המקיפות ורבים בגוף האדם כמו הגלגלת יקיפהו שטח אחד וכן אברים רבים והגוף יקרא אחד | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | === It is similar to an odd number === | ||
| + | |||
| + | |style="text-align:right;"|[[ספר_המספר_/_אברהם_אבן_עזרא#similar_to_an_odd_number|<span style=color:blue>'''והוא דומה לנפרד'''</span>]] | ||
| + | |- | ||
| + | |Explanation: when you sum up the odds in sequence the squares of the number of the odds are generated. | ||
| + | |style="text-align:right;"|פי' שכשתחבר הנפרדים על הסדר יולידו מרובעי מספר הנפרדים | ||
| + | |- | ||
| + | |Because 1 is the square of 1. | ||
| + | :<math>\scriptstyle{\color{blue}{1=1^2}}</math> | ||
| + | |style="text-align:right;"|כי א' הוא מרובע א‫' | ||
| + | |- | ||
| + | |1 and [3] is the square of 2. | ||
| + | :<math>\scriptstyle{\color{blue}{1+3=2^2}}</math> | ||
| + | |style="text-align:right;"|וא' עם הוא מרובע ב‫' | ||
| + | |- | ||
| + | |1, 3 and 5 is the square of 3. | ||
| + | :<math>\scriptstyle{\color{blue}{1+3+5=3^2}}</math> | ||
| + | |style="text-align:right;"|וא' עם ג' וה' הוא מרובע ג‫' | ||
| + | |- | ||
| + | |This is one of the properties of the number. | ||
| + | |style="text-align:right;"|וזה אחד מסגלת המספר | ||
| + | |- | ||
| + | | | ||
| + | === It is a half of an eighth === | ||
| + | |||
| + | |style="text-align:right;"|[[ספר_המספר_/_אברהם_אבן_עזרא#half_of_an_eighth|<span style=color:blue>'''הוא חצי שמינית'''</span>]] | ||
| + | |- | ||
| + | |If you divide the product by the square of the denominator, the result of division is an integer. | ||
| + | |style="text-align:right;"|אם תחלק הנכפל על מרובע המורה יהיה היוצא מהחלוקה שלמים | ||
| + | |- | ||
| + | |If it cannot be divided, i.e. the square of the denominator is greater than the product, or it can be divided, but there is a remainder that cannot be divided, they are fractions named by the name of the square. | ||
| + | |style="text-align:right;"|ואם לא יתחלק ר"ל ‫<ref>77v</ref>שיהיה מרובע המורה יותר גדול מהנכפל או יתחלק וישאר שלא יתחלק יהיו שברים נקראין בשם המרובע | ||
| + | |- | ||
| + | |If you divide the product by the denominator, the result are fractions named by the name of the denominator and what cannot be divided are fractions of fractions named by the name of the denominator. Understand. | ||
| + | |style="text-align:right;"|ואם תחלק הנכפל על המורה יהיה היוצא שברים נקראין בשם המורה ומה שלא יתחלק יהיו שברי שברים נקראין בשם המורה ובין | ||
| + | |- | ||
| + | |Let this rule be handed down to you: after you know the denominator, its square, and the product, divide the square by the product, or divide the product by the denominator. | ||
| + | |style="text-align:right;"|ויהיה כלל זה מסור בידך אחר שתדע המורה ומרובעו והנכפל תחלק המרובע על הנכפל או תחלק הנכפל על המורה | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | === But I shall teach you a short method === | ||
| + | |||
| + | |style="text-align:right;"|[[ספר_המספר_/_אברהם_אבן_עזרא#short_method|<span style=color:blue>'''רק אלמד דרך קצרה'''</span>]] | ||
| + | |- | ||
| + | |Explanation: because according to what we said above, one denominator should be produced for all of them by multiplying one by the other, because the reason for the denominator issue is to find a number that will have all of these parts. | ||
| + | |style="text-align:right;"|פי' כי לפי מה שאמרנו למעלה היה צריך לעשות מורה אחד לכלן בכפל האחד על האחר כי הסבה מענין המורה הוא למצא מספר שיהיו בו כל חלקים אלו | ||
| + | |- | ||
| + | | | ||
| + | :He did not really need to take sixths, because the thirds were enough for him: by multiplying [3] by 5; it is 15. We multiply it by 7; it is 105. We multiply it by 8; it is 840 and this is the common denominator. | ||
| + | :<math>\scriptstyle{\color{blue}{3\sdot5\sdot7\sdot8=15\sdot7\sdot8=105\sdot8=840}}</math> | ||
| + | |style="text-align:right;"|עוד באמת לא היה צריך לקחת ששיים כי די לו בשלישיות וזה נכפול על ה' יהיו ט"ו עוד נכפול זה על ז' יהיו ק"ה עו' נכפול זה בח' יהיו תק"מ והוא המורה | ||
| + | |- | ||
| + | | | ||
| + | :We look for the first number which is 2-thirds of a quarter of a fifth; it is 28. | ||
| + | |style="text-align:right;"|נבקש המספר ראשון שהוא ב' שלישית רביעית החמשית יהיו כ"ח | ||
| + | |- | ||
| + | | | ||
| + | :Because the fifth is 168. | ||
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{5}\sdot840=168}}</math> | ||
| + | |style="text-align:right;"|כי החמשית הוא קס"ח | ||
| + | |- | ||
| + | | | ||
| + | :Its quarter is 42. | ||
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{4}\sdot168=42}}</math> | ||
| + | |style="text-align:right;"|ורביעיתו מ"ב | ||
| + | |- | ||
| + | | | ||
| + | :Its two-thirds are 28 and this is the first number. | ||
| + | :<math>\scriptstyle{\color{blue}{\frac{2}{3}\sdot42=28}}</math> | ||
| + | |style="text-align:right;"|ושתי שלישיותיו כ"ח וזהו החשבון הראשון | ||
| + | |- | ||
| + | | | ||
| + | :We know that its eighth is 105. | ||
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{8}\sdot840=105}}</math> | ||
| + | |style="text-align:right;"|וידענו ששמיניתו ק"ה | ||
| + | |- | ||
| + | | | ||
| + | :Its seventh is 15. | ||
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{7}\sdot105=15}}</math> | ||
| + | |style="text-align:right;"|ושביעיתו ט"ו | ||
| + | |- | ||
| + | | | ||
| + | :Its six-sevenths are 90. | ||
| + | :<math>\scriptstyle{\color{blue}{\frac{6}{7}\sdot105=90}}</math> | ||
| + | |style="text-align:right;"|והשש שביעיות הם צ‫' | ||
| + | |- | ||
| + | | | ||
| + | :We multiply 28 by 90; the result is two thousand and 5[2]0. | ||
| + | |style="text-align:right;"|נכפול כ"ח על צ' יעלה אלפים ת"ק | ||
| + | |- | ||
| + | | | ||
| + | :We divide it by 840, which is the denominator; the result is 3 and they are parts, of which 840 are one unit, which are a fifth of a seventh of an eighth, the eighth [of 840] is 105, its seventh is 15 and its fifth is 3 and this is the required. | ||
| + | :<math>\scriptstyle{\color{blue}{\frac{28\sdot90}{840}=\frac{2520}{840}=3}}</math> | ||
| + | |style="text-align:right;"|חלקנום על תת"מ שהוא המורה ויעלה ג' שהן חלקים מהתת"מ שלמים והן חמשית שביעית השמינית שהשמינית ק"ה ושביעיתו ט"ו וחמישיתו ג' והוא הדרוש | ||
| + | |- | ||
| + | |It is necessary to examine the example that the scholar chose, because it is more appropriate to take the example with the smallest possible number. | ||
| + | |style="text-align:right;"|וצ"ע בדמיון שלקח החכם כי יותר ראוי לקחת הדמיון בקטן המספר שאפשר | ||
| + | |- | ||
| + | |It is also necessary to examine why it was necessary to explain that since we have sixths, there is no need for a third as if the sixth had to be divided. | ||
| + | |style="text-align:right;"|גם צ"ע למה הוצרך לבאר שאחר שיש לנו ששיות אין צריך לשלישית כאלו היה צריך לחלק ששית | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | === The difference between the fifth and the ninth is four === | ||
| + | |||
| + | |style="text-align:right;"|[[ספר_המספר_/_אברהם_אבן_עזרא#difference_between_fifth_and_ninth|<span style=color:blue>'''החמשית והתשיעית ארבע'''</span>]] | ||
| + | |- | ||
| + | |It is necessary to examine how he used the first method, because it does not apply to all numbers: | ||
| + | |style="text-align:right;"|צ"ע איך לקח דרך זה הראשון כי לא ימשך בכל המספרים | ||
| + | |- | ||
| + | | | ||
| + | *This is when we say: we take a third of the amount, plus its fifth and its seventh. How much is it from the value of the amount? | ||
| + | :<math>\scriptstyle\frac{1}{3}+\frac{1}{5}+\frac{1}{7}</math> | ||
| + | |style="text-align:right;"|וזה שנאמר לקחנו שלישית הממון וחמשיתו ושביעיתו<br> | ||
| + | כמה הוא מערך הממון | ||
| + | |- | ||
| + | | | ||
| + | :According to his way it is as follows: | ||
| + | |style="text-align:right;"|ולפי דרכו יהיה כן | ||
| + | |- | ||
| + | | | ||
| + | :We consider them as three sevenths, which is the smallest fraction. | ||
| + | |style="text-align:right;"|נחשב כאלו הן ג' שביעיות שהוא השבר היותר פחות אחר | ||
| + | |- | ||
| + | | | ||
| + | :We multiply what is between the seventh and the third, which is 4, by what is between the fifth and the third, which is 2; the result of the multiplication is 8. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(\frac{1}{3}-\frac{1}{7}\right)\sdot\left(\frac{1}{3}-\frac{1}{5}\right)=\frac{4}{3\sdot7}\sdot\frac{2}{3\sdot5}=\frac{8}{3\sdot7\sdot3\sdot5}}}</math> | ||
| + | |style="text-align:right;"|נכפול מה שבין השביעית והשלישית ‫<ref>78r</ref>שהוא ד' על מה שבין החמישית והשלישית שהוא ב' ויעלה הכפל ח‫' | ||
| + | |- | ||
| + | | | ||
| + | :We convert five [of the 8] to a seventh; they are 4-sevenths and 3 remains [of the 8]. | ||
| + | :<math>\scriptstyle{\color{blue}{\frac{8}{3\sdot7\sdot3\sdot5}=\frac{1}{3\sdot7\sdot3}=\frac{3}{3\sdot7\sdot3\sdot5}}}</math> | ||
| + | |style="text-align:right;"|ונעשה מהחמשה שביעית אחת ויהיו ד' שביעיות וישאר ג‫' | ||
| + | |- | ||
| + | | | ||
| + | :We add it to 2, which is the difference between the third and the fifth; it is 3. | ||
| + | |style="text-align:right;"|ונחברם עם ב' שהוא היתרון שבין שלישית לחמישית והיה ה‫' | ||
| + | |- | ||
| + | | | ||
| + | :According to this the ratio is 5-sevenths; the result is 75, since the denominator is 105. | ||
| + | |style="text-align:right;"|ולפי זה יהיה הערך ה' שביעיות ויעלה ע"ה לפי שהמורה ק"ה | ||
| + | |- | ||
| + | |colspan="2" | | ||
| + | :<span style=color:green>It seems that the procedure described is as follows:</span> | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\begin{align}\scriptstyle\frac{1}{3}+\frac{1}{5}+\frac{1}{7}&\scriptstyle=\frac{3}{7}+\left(\frac{1}{5}-\frac{1}{7}\right)+\left(\frac{1}{3}-\frac{1}{7}\right)=\frac{3}{7}+\frac{\frac{2}{5}}{7}+\frac{\frac{4}{3}}{7}=\frac{3}{7}+\frac{\frac{6}{5}-\frac{4}{5}}{7}+\frac{\frac{4}{3}}{7}\\&\scriptstyle=\frac{3}{7}+\frac{\frac{6}{5}}{7}+\frac{4\sdot\left(\frac{1}{3}-\frac{1}{5}\right)}{7}=\frac{3}{7}+\frac{1}{7}+\frac{\frac{1}{5}}{7}+\frac{\frac{4\sdot\frac{2}{3}}{5}}{7}\\\end{align}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :According to the second way, which is the appropriate one, it is 7[1] parts of [10]5. | ||
| + | |style="text-align:right;"|ולפי הדרך שני שהוא האמתי יהיו ע"ה חלקים מן ה‫' | ||
| + | |- | ||
| + | |It is also necessary to examine how he said in his example that the three are 3-fifths of a seventh of a ninth, when he initially said: we convert the 6 into a ninth. | ||
| + | |style="text-align:right;"|עו' צ"ע איך אמ' בדמיונו שהשלשה היו ג' חמשיות שביעית התשיעית והוא אמר תחלה נעשה מן הו' תשיעית אחת | ||
| + | |- | ||
| + | |There is another correct way to do this, only the increment between the [denominators of the] fractions is the same, such as 4, 5, 6, or 5, 7, 9, and it is to take the type of the middle fraction, instead of the three types of the three fractions, then the difference between the extremes is multiplied by the increment, and the sum is the required. | ||
| + | |style="text-align:right;"|ויש בזה דרך אחרת נכונה רק שיהיה הדלוג בשברים שוה כמו ד'ה'ו' ה'ז'ט' והוא לקחת מקום הג' מינין ג' שברים ממין האמצעי ואחר יוכפל מרחק הקצוות כמרחק הדלוגים והמחובר הוא הדרוש | ||
| + | |- | ||
| + | |colspan="2" | | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\frac{1}{n}+\frac{1}{n+d}+\frac{1}{n+2d}=\frac{3}{n+d}+\frac{\left(\frac{1}{n}-\frac{1}{n+2d}\right)\sdot d}{n+d}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | === Example: The ratio of 4 to [6] === | ||
| + | |||
| + | |style="text-align:right;"|[[ספר_המספר_/_אברהם_אבן_עזרא#ratio_of_4_6_8|<span style=color:blue>'''דמיון ערך ד' וה‫''''</span>]] | ||
| + | |- | ||
| + | | | ||
| + | :This is clear, because the square of the entire line, which is 16, is equal to the two squares of 12 and 4 in the proposition 4 of Euclid's II. | ||
| + | |style="text-align:right;"|פי' וזה יתבאר כי מרובע הקו כלו שהוא י"ו שוה לשני מרובעי י"ב וד' בתמונת ד' מב' לאקלידיס | ||
| + | |- | ||
| + | | | ||
| + | :The product of 6 by 8 is equal to the surface enclosed by 12 and 4. | ||
| + | :<math>\scriptstyle{\color{blue}{6\times8=12\times4}}</math> | ||
| + | |style="text-align:right;"|ושטח ו' בח' שוה לשטח שיקיפו בו י"ב וד‫' | ||
| + | |- | ||
| + | | | ||
| + | :Since the ratio of 4 to 6 is the same as the ratio of 8 to 12. | ||
| + | :<math>\scriptstyle{\color{blue}{4:6=8:12}}</math> | ||
| + | |style="text-align:right;"|לפי שיחס ד' אל ו' כיחס ח' אל י"ב | ||
| + | |- | ||
| + | | | ||
| + | :Therefore, the square of 16 is equal to the two squares of 12 and 4 and double the surface enclosed by 6 and 8. | ||
| + | :<math>\scriptstyle{\color{blue}{16^2=12^2+4^2+2\sdot\left(6\times8\right)}}</math> | ||
| + | |style="text-align:right;"|אם כן מרובע י"ו שוה לשני מרובעי י"ב וד' ולכפל השטח שיקיפו בו ו' וח‫' | ||
| + | |- | ||
| + | | | ||
| + | :But [the sum of] the two squares of 6 and 8 is equal to the double the surface enclosed by 6 and 8, with the square of [the difference] between 6 and 8, as explained in II.5. | ||
| + | :<math>\scriptstyle{\color{blue}{6^2+8^2=2\sdot\left(6\times8\right)+\left(8-6\right)^2}}</math> | ||
| + | |style="text-align:right;"|אבל שני מרובעי ו' ח' שוין לכפל השטח שיקיפו בו ו' ח' עם מרובע מה שבין ו' ח' כמבואר בה' מב‫' | ||
| + | |- | ||
| + | | | ||
| + | :Therefore, the square of 16 with the square of the difference between 6 and 8 is equal to the four squares of 4, 6, 8 and 12. This is explained from the second and third. | ||
| + | :<math>\scriptstyle{\color{blue}{16^2+\left(8-6\right)^2=4^2+6^2+8^2+12^2}}</math> | ||
| + | |style="text-align:right;"|אם כן מרובע י"ו עם מרובע היתרון שבין ו' לח' שוה לד' מרובעי ד' ו' ח' י"ב ובזה יתבאר מהשני והשלישי | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | === Its proportions are composed === | ||
| + | |||
| + | |style="text-align:right;"|[[ספר_המספר_/_אברהם_אבן_עזרא#its_proportions_are_composed|<span style=color:blue>'''כי ערכיה מורכבין'''</span>]] | ||
| + | |- | ||
| + | |Explanation: the harmonic proportions consist of the geometric and the arithmetic proportions, as they are determined by the division of the excess of the one by the other and the ratio of one number by the other number. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\left(a_2-a_1\right):\left(a_3-a_2\right)=a_1:a_3}}</math> | ||
| + | |style="text-align:right;"|פי' ערכי הנגונים הן מורכבים ערכי המדות וערכי המספר כי יעשה בחלק היתרון האחד על האחר וכיחס המספר האחד אל המספר האחר | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | === Because the ratio is always === | ||
| + | |||
| + | |style="text-align:right;"|[[ספר_המספר_/_אברהם_אבן_עזרא#ratio_between_first_and_last|<span style=color:blue>'''כי לעולם יהיה הערך'''</span>]] | ||
| + | |- | ||
| + | |Because the ratio of what is between the first and the mean to what is between the mean and the last is always the same as the ratio of the first number to the last number. | ||
| + | |style="text-align:right;"|כי לעולם יהיה היחס מה שבין הראשון והאמצעי אל מה שבין האמצעי והאחרון כיחס המספר הראשון אל האחרון | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | === Question: Reuven hired Shimon === | ||
| + | |||
| + | |style="text-align:right;"|[[ספר_המספר_/_אברהם_אבן_עזרא#Reuven_hired_Shimon|<span style=color:blue>'''שאלה ראובן שכר שמעון'''</span>]] | ||
| + | |- | ||
| + | | | ||
| + | :The rule of all these methods is that we multiply 11 by 9; it is 99. Divide it by 17; the result is 5 pešuṭim and 14 parts of 17 of one pašuṭ. | ||
| + | :<math>\scriptstyle{\color{blue}{\frac{11\sdot9}{17}=\frac{99}{17}=5+\frac{14}{17}}}</math> | ||
| + | |style="text-align:right;"|הכלל בכל דרכים אלו שנכפול י"א על ט' ויהיו צ"ט וחלקם על י"ז ועלה ה' פשו' וי"ד חלקים מי"ז ‫<ref>78v</ref>בפשו‫' | ||
| + | |- | ||
| + | |This rule is given to you in proportions: the number that corresponds to the unknown, which is in the place of the zero, is the denominator. If zero is one of the extremes, the other extreme is the denominator; and if zero is one of the means, the other mean is the denominator. | ||
| + | |style="text-align:right;"|כלל זה יהיה בידך בערכין שאותו המספר שיהיה גיל הנעלם שהוא מקום הגלגל מן המורה שאם היה הגלגל אחד מהקצוות הקצה האחר הוא המורה ואם הגלגל הוא אחד מהאמצעיים האמצעי האחר הוא המורה | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | === And you do not know the third: multiply the first by the second === | ||
| + | |||
| + | |style="text-align:right;"|[[ספר_המספר_/_אברהם_אבן_עזרא#the_third_is_unknown|<span style=color:blue>'''ולא תדע השלישי כפול הראשון על השני'''</span>]] | ||
| + | |- | ||
| + | |This is clear from 19.V: when the sum to the sum is as the ratio of the whole to the whole, then the ratio of the remainder to the remainder is as the ratio of the whole to the whole. | ||
| + | |style="text-align:right;"|זה יתבאר מי"ט מה' באמת כאשר היה המחובר אל המחובר כיחס הכל אל הכל הנה יחס הנשאר אל הנשאר כיחס הכל אל הכל | ||
| + | |- | ||
| + | | | ||
| + | :Therefore, in his second example, since the ratio of the unknown difference between 3 and 4 to the difference between 4 and the unknown number is as the ratio of the known 3 to the unknown 6, and the unknown is greater than its excess over 4, then 3 is greater than the difference between 3 and 4, according to Euclid's 14.V. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(4-3\right):\left(6-4\right)=3:6}}</math> | ||
| + | :<math>\scriptstyle{\color{blue}{6>\left(6-4\right)}}</math> | ||
| + | :<math>\scriptstyle{\color{blue}{3>\left(4-3\right)}}</math> | ||
| + | |style="text-align:right;"|ולכן בדמיונו השני לפי שיחס היתרון שבין ג' וד' הידוע אל היתרון שבין ד' והמספר הנעלם כיחס ג' הידוע אל ו' הנעלם והנה הנעלם גדול מיתרונו על ד' הנה אם כן ג' גדול יותר מהיתרון שבין ג' לד' מי"ד מה' אקלידיס | ||
| + | |- | ||
| + | | | ||
| + | :The difference between 3 and 4 is known, so when we subtract it from 3, the remainder is known and it is 2. | ||
| + | :<math>\scriptstyle{\color{blue}{3-\left(4-3\right)=2}}</math> | ||
| + | |style="text-align:right;"|והיתרון שבין ג' לד' ידוע אם כן כשחסרנוהו מג' יהיה הנשאר ידוע והוא ב‫' | ||
| + | |- | ||
| + | | | ||
| + | :We also know that 4 is the remainder, when we subtract from 6 the excess of 6 over it. | ||
| + | :<math>\scriptstyle{\color{blue}{6-\left(6-4\right)=4}}</math> | ||
| + | |style="text-align:right;"|וגם כן ידענו שד' הוא הנשאר כשחסרנו מו' היתרון שנוסף על ו‫' | ||
| + | |- | ||
| + | | | ||
| + | :Now, we have three known numbers: | ||
| + | |style="text-align:right;"|ועתה יש לנו ג' מספרים ידועין | ||
| + | |- | ||
| + | | | ||
| + | :The first is 2 and it is the remainder from 3, when the difference between 3 and 4 is subtracted from it. | ||
| + | :<math>\scriptstyle{\color{blue}{a_1=3-\left(4-3\right)=2}}</math> | ||
| + | |style="text-align:right;"|האחד ב' והוא הנשאר מג' כשחוסר ממנו היתרון שבין ג' לד‫' | ||
| + | |- | ||
| + | | | ||
| + | :The second is 3, which is the known whole. | ||
| + | :<math>\scriptstyle{\color{blue}{a_2=3}}</math> | ||
| + | |style="text-align:right;"|השני ג' שהוא הכל ידוע | ||
| + | |- | ||
| + | | | ||
| + | :The third is 4, which is the remainder from the unknown, when its excess over 4 is subtracted from it. | ||
| + | :<math>\scriptstyle{\color{blue}{a_3=a-\left(a-4\right)=4}}</math> | ||
| + | |style="text-align:right;"|השלישי ד' שהוא הנשאר מהנעלם כשחוסר ממנו היתרון שהוא נוסף על ד‫' | ||
| + | |- | ||
| + | | | ||
| + | :We know that the ratio of the subtracted from 3 to the subtracted from the unknown is as the ratio of 3, which is the whole, to the unknown. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(4-3\right):\left(a-4\right)=3:a}}</math> | ||
| + | |style="text-align:right;"|וידענו שיחס המחוסר מג' אל המחוסר מהנעלם כיחס ג' שהוא הכל אל הנעלם | ||
| + | |- | ||
| + | | | ||
| + | :Therefore, the ratio of 2, which is the remainder, to 4, which is the remainder, is as the ratio of 3 to the unknown. | ||
| + | :<math>\scriptstyle{\color{blue}{\left[3-\left(4-3\right)\right]:\left[a-\left(a-4\right)\right]=2:4=3:a}}</math> | ||
| + | |style="text-align:right;"|אם כן יחס ב' הנשאר אל ד' הנשאר כיחס ג' אל הנעלם | ||
| + | |- | ||
| + | | | ||
| + | :We multiply the means, which are 4 and 3; it is 12. | ||
| + | |style="text-align:right;"|ולכן נכפול האמצעיים שהן ד' וג' והיו י"ב | ||
| + | |- | ||
| + | | | ||
| + | :We divide 12 by 2; it is 6, etc. | ||
| + | :<math>\scriptstyle{\color{blue}{a_4=a=\frac{a_2\sdot a_3}{a_1}=\frac{3\sdot4}{2}=\frac{12}{2}=6}}</math> | ||
| + | |style="text-align:right;"|ונחלק י"ב על ב' והיה ו' וכו‫' | ||
| + | |- | ||
| + | |Understand the others from Euclid's 18.V. | ||
| + | |style="text-align:right;"|וכן תבין האחרים מי"ח מה' אקלידיס | ||
| + | |- | ||
| + | |Proposition 16 is derived this way: the first and the seventh are taken, and they are cardinal points. One proposition is derived from both of them. From the fourth and tenth, which are cardinal points, another proposition is derived. Then, from both of them, one proposition is derived, called the 16th, and is the strongest of them all. | ||
| + | |style="text-align:right;"|צורת הי"ו מוציאין אותה בדרך זו לוקחין הראשונה והשביעית והן יתדות ומוציאין משתיהן צורה אחת ומהרביעית והעשירית והן יתדות מוציאין צורה אחרת ומשתיהן מוציאין צורה אחת והיא נקראת הי"ו והיא חזקה מכלן | ||
| + | |- | ||
| + | |I have found, with the help of God, proposition 16: We take the first and seventh propositions and derive one proposition from both of them. We take proposition 15, and from these two, we derive another proposition, which is the 16th, and this is a secret. | ||
| + | |style="text-align:right;"|ומצאתי בע"ה צורת הי"ו נקח הצורה הראשונה והשביעית ועושין משתיהן צורה אחת ונקח צורת הט"ו ‫<ref>79r</ref>ומשתים אלו נעשה צורה אחרת והיא הי"ו וזה סוד | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | == Additional Word Problems (P1026) == | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | *Question: three went to the market to buy a fish, whose price is 5 pešuṭim. | ||
| + | :One said: I will give everything in my purse and you will give only half of what is in your purse. | ||
| + | :Another said: I will give everything in my purse and you will give only a third of what is in your purse. | ||
| + | :Another said: I will give everything in my purse and you will give only a quarter of what is in your purse. | ||
| + | :How much is in the purse of each of them? | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle a+\frac{1}{2}\sdot\left(b+c\right)=5\\\scriptstyle b+\frac{1}{3}\sdot\left(a+c\right)=5\\\scriptstyle c+\frac{1}{4}\sdot\left(a+b\right)=5\end{cases}</math> | ||
| + | |style="text-align:right;"|<big>שאלה</big> שלשה הלכו לקנות דג בשוק ערכו ה' פשו‫'<br> | ||
| + | אמר אחד אני אתן כל מה שבכיסי ואתם לא תתנו רק החצי שבכיסכם<br> | ||
| + | אמר האחר אני אתן כל מה שבכיסי ואתם לא תתנו רק שליש שלכם<br> | ||
| + | אמר האחר אני אתן כל מה שבכיסי ואתם לא תתנו רק הרביע שבכיסכם<br> | ||
| + | כמה יהיה בכיס כל אחד | ||
| + | |- | ||
| + | | | ||
| + | ::We look for the common denominator; it is 12. | ||
| + | |style="text-align:right;"|נבקש המורה והוא י"ב | ||
| + | |- | ||
| + | | | ||
| + | ::29: we can extract three numbers from it, so that the three [sums] will be equal to the same number: 5, 11, 13. | ||
| + | |style="text-align:right;"|כ"ט כי ממנו נוכל לעשות ג' מספרים שישתוו שלשתן למספר אחד והן ה' י"א י"ג | ||
| + | |- | ||
| + | | | ||
| + | ::The owner of the 5 will ask for a half. | ||
| + | ::<math>\scriptstyle{\color{OliveGreen}{5+\frac{1}{2}\sdot\left(11+13\right)}}</math> | ||
| + | |style="text-align:right;"|בעל ה' ישאל החצי | ||
| + | |- | ||
| + | | | ||
| + | ::The owner of the 11 will ask for a third. | ||
| + | ::<math>\scriptstyle{\color{OliveGreen}{11+\frac{1}{3}\sdot\left(5+13\right)}}</math> | ||
| + | |style="text-align:right;"|בעל י"א ישאל השליש | ||
| + | |- | ||
| + | | | ||
| + | ::The owner of the 13 will ask for a quarter. | ||
| + | ::<math>\scriptstyle{\color{OliveGreen}{13+\frac{1}{4}\sdot\left(5+11\right)}}</math> | ||
| + | |style="text-align:right;"|בעל י"ג ישאל הרביע | ||
| + | |- | ||
| + | | | ||
| + | *Question: Reuven, Shimon, Levi and Yehuda. | ||
| + | :Reuven told Shimon: everything in my purse plus a half of what is in your purse is 5 dinar. | ||
| + | :Shimon told Levi: everything in my purse plus a third of what is in your purse is 5 dinar. | ||
| + | :Levi told Yehuda: everything in my purse plus a quarter of what is in your purse is 5 dinar. | ||
| + | :Yehuda, the fourth, told Reuven, the first: everything in my purse plus a fifth of what is in your purse is 5 dinar. | ||
| + | :How much is in the purse of each of them? | ||
| + | |style="text-align:right;"|<big>שאלה</big> ראובן שמעון לוי ויהודה<br> | ||
| + | אמ' ראובן לשמעון כל מה שבכיסי עם חצי שבכיסך הוא ה' די‫'<br> | ||
| + | אמ' שמעון ללוי כל מה שבכיסי עם שליש שבכיסך הוא ה' די‫'<br> | ||
| + | אמ' לוי ליהודה כל מה שבכיסי עם רביע מה שבכיסך הוא ה' די‫'<br> | ||
| + | אמ' יהודה הרביעי לראובן הראשון כל מה שבכיסי עם חומש מה שבכיסך הוא ה' די‫'<br> | ||
| + | כמה בכיס כל אחד | ||
| + | |- | ||
| + | | | ||
| + | :The answer: | ||
| + | ::Reuven: 37 pešuṭim and 97 parts of 11[9] of one pašuṭ | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{37}{12}+\frac{\frac{97}{119}}{12}}}</math> | ||
| + | |style="text-align:right;"|<big>תשובה</big> ראובן ל"ז פשו' צ"ז חלקי' מקי"ו בפשו‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Shimon: 44 pešiṭim and 44 parts. | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{44}{12}+\frac{\frac{44}{119}}{12}}}</math> | ||
| + | |style="text-align:right;"|שמעון מ"ד פשי' מ"ד חלקים | ||
| + | |- | ||
| + | | | ||
| + | ::Levi: 46 pešuṭim and 106 parts. | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{46}{12}+\frac{\frac{106}{119}}{12}}}</math> | ||
| + | |style="text-align:right;"|לוי מ"ו פשו' ק"ו חלקים | ||
| + | |- | ||
| + | | | ||
| + | ::Yehuda: 52 pešiṭim and 52 parts. | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{52}{12}+\frac{\frac{52}{119}}{12}}}</math> | ||
| + | |style="text-align:right;"|יהודה נ"ב פשי' נב חלקים | ||
| + | |- | ||
| + | | | ||
| + | ::We find that all that Reuven has with half of what Shimon has is 5. | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(\frac{37}{12}+\frac{\frac{97}{119}}{12}\right)+\frac{1}{2}\sdot\left(\frac{44}{12}+\frac{\frac{44}{119}}{12}\right)}}</math> | ||
| + | |style="text-align:right;"|ובזה נמצא כל ראובן עם חצי שמעון ה‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Also what Shimon has with a third of what Levi has. | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(\frac{44}{12}+\frac{\frac{44}{119}}{12}\right)+\frac{1}{3}\sdot\left(\frac{46}{12}+\frac{\frac{106}{119}}{12}\right)}}</math> | ||
| + | |style="text-align:right;"|וכן שמעון עם שליש לוי | ||
| + | |- | ||
| + | | | ||
| + | ::Also what Levi has with a quarter of what Yehuda has. | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(\frac{46}{12}+\frac{\frac{106}{119}}{12}\right)+\frac{1}{4}\sdot\left(\frac{52}{12}+\frac{\frac{52}{119}}{12}\right)}}</math> | ||
| + | |style="text-align:right;"|וכן לוי עם רביע יהודה <s>אם כן ראובן שוה</s> | ||
| + | |- | ||
| + | | | ||
| + | ::Also what Yehuda has with a fifth of what Reuven has. | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(\frac{52}{12}+\frac{\frac{52}{119}}{12}\right)+\frac{1}{5}\sdot\left(\frac{37}{12}+\frac{\frac{97}{119}}{12}\right)}}</math> | ||
| + | |style="text-align:right;"|וכן יהודה עם חומש ראובן | ||
| + | |- | ||
| + | | | ||
| + | ::We subtract the common half of Shimon; what Reuven has remains equal to half of what Shimon has with a third of what Levi has. | ||
| + | |style="text-align:right;"|נסיר חצי שמעון המשותף וישאר ראובן שוה לחצי שמעון עם שליש לוי | ||
| + | |- | ||
| + | | colspan=2| | ||
| + | ::<math>\scriptstyle{\color{blue}{Reuven+\frac{1}{2}\sdot Shimon=Shimon+\frac{1}{3}\sdot Levi\quad/-\frac{1}{2}\sdot Shimon}}</math> | ||
| + | ::<math>\scriptstyle{\color{blue}{Reuven=\frac{1}{2}\sdot Shimon+\frac{1}{3}\sdot Levi}}</math> | ||
| + | |- | ||
| + | | | ||
| + | ::What Shimon has is equal to two-thirds of what Levi has with a quarter of what Yehuda has. | ||
| + | |style="text-align:right;"|ושמעון שוה לשני שלישי לוי עם רביע יהודה | ||
| + | |- | ||
| + | | colspan=2| | ||
| + | ::<math>\scriptstyle{\color{OliveGreen}{Shimon+\frac{1}{3}\sdot Levi=Levi+\frac{1}{4}\sdot Yehuda\quad/-\frac{1}{3}\sdot Levi}}</math> | ||
| + | ::<math>\scriptstyle{\color{blue}{Shimon=\frac{2}{3}\sdot Levi+\frac{1}{4}\sdot Yehuda}}</math> | ||
| + | |- | ||
| + | | | ||
| + | ::So, what Reuven has is equal to two-thirds of what Levi has with an eighth of what Yehuda has. | ||
| + | |style="text-align:right;"|אם כן ראובן שוה לשני שלישי לוי עם שמינית יהודה | ||
| + | |- | ||
| + | | colspan=2| | ||
| + | ::<math>\scriptstyle{\color{blue}{Reuven=\frac{2}{3}\sdot Levi +\frac{1}{8}\sdot Yehuda}}</math> | ||
| + | |- | ||
| + | | | ||
| + | ::What Levi has is equal to 3-quarters of what Yehuda has with a fifth of what Reuven has. | ||
| + | |style="text-align:right;"|אם כן לוי שוה לג' רבעי יהודה עם חומש ראובן | ||
| + | |- | ||
| + | | colspan=2| | ||
| + | ::<math>\scriptstyle{\color{OliveGreen}{Levi+\frac{1}{4}\sdot Yehuda=Yehuda+\frac{1}{5}\sdot Reuven\quad/-\frac{1}{4}\sdot Yehuda}}</math> | ||
| + | ::<math>\scriptstyle{\color{blue}{Levi=\frac{3}{4}\sdot Yehuda+\frac{1}{5}\sdot Reuven}}</math> | ||
| + | |- | ||
| + | | | ||
| + | ::So, what Reuven has is equal to 5-eighths of what Yehuda has with 2 parts of 15 of what Reuven him self has. | ||
| + | |style="text-align:right;"|אם כן ראובן שוה לה' שמיניות יהודה וב' חלקי' מט"ו מראובן עצמו | ||
| + | |- | ||
| + | | colspan=2| | ||
| + | ::<math>\scriptstyle{\color{blue}{Reuven=\frac{5}{8}\sdot Yehuda+\frac{2}{15}\sdot Reuven}}</math> | ||
| + | |- | ||
| + | | | ||
| + | ::Reuven has 75; Shimon has 88; Levi has 93; Yehuda has 104 | ||
| + | |style="text-align:right;"|א'פ'ד' ראובן ע"ה שמעון פ"ח לוי צ"ג יהודה ק"ד | ||
| + | |- | ||
| + | | | ||
| + | ::Or, Reuven has 37 and a half; Shimon has 44; Levi has 46 and a half; Yehuda has 52 | ||
| + | |style="text-align:right;"|או ראובן ל"ז וחצי ‫<ref>79v</ref>שמעון מ"ד לוי מ"ו וחצי יהודה נ"ב | ||
| + | |- | ||
| + | | | ||
| + | ::And so on for their halves. | ||
| + | |style="text-align:right;"|וכן לחציין וכן וכן | ||
| + | |- | ||
| + | |Question: a man arrived to a country and took an oath that if he will double his money every day, he will would spend a certain amount of it each day. After a few days he had nothing left. | ||
| + | :How much money did he have? | ||
| + | |style="text-align:right;"|<big>שאלה</big> אדם נכנס [בע]יר ונודר שאם יכפול ממונו בכל יום יוציא ממנו בכל יום סך מוגבל ולימים לא נשאר בידו מאומה<br> | ||
| + | כמה הוא הממון שבידו | ||
| + | |- | ||
| + | |The method: we look for a number, such that, when doubled in a double ratio as many times as the number of days, at the end of which he has nothing left, the result is the amount he spends every day. We subtract this required number from the amount he spends each day and the remainder is the amount of money he has. | ||
| + | |style="text-align:right;"|<big>הדרך</big> בזה שנבקש מספר שבהכפלו פעמים כמספר הימים על יחס הכפל שאחריהם לא נשאר לו מאומה יעלה לסך שיוציא מהם בכל יום והמספר ההוא המבוקש נגרע מהסך שיוציא בכל יום והנשאר הוא הממון שבידו | ||
| + | |- | ||
| + | | | ||
| + | :*Example: he spends 48 pešiṭim every day and at the end of three days he has nothing left. | ||
| + | |style="text-align:right;"|<big>המשל</big> שיוציא בכל יום מ"ח פשי' ולסוף ג' ימים לא נשאר לו מאומה | ||
| + | |- | ||
| + | | | ||
| + | ::6 is the number that when doubled in a double ratio as many times as the number of days, which is 3, the result is 48. | ||
| + | |style="text-align:right;"|הנה המספר שבהכפלו על יחס הכפל כמספר הימים שהם ג' יעלה מ"ח הוא ו‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Because, when we double 6, the result is 12. | ||
| + | ::<math>\scriptstyle{\color{blue}{2\sdot6=12}}</math> | ||
| + | |style="text-align:right;"|כי כשנכפול ו' יעלה י"ב | ||
| + | |- | ||
| + | | | ||
| + | ::When we double 12, the result is 24. | ||
| + | ::<math>\scriptstyle{\color{blue}{2\sdot12=24}}</math> | ||
| + | |style="text-align:right;"|וכשנכפול י"ב עלה כ"ד | ||
| + | |- | ||
| + | | | ||
| + | ::And when we double 24, the result is 48. | ||
| + | ::<math>\scriptstyle{\color{blue}{2\sdot24=48}}</math> | ||
| + | |style="text-align:right;"|וכשנכפול כ"ד עלה מ"ח | ||
| + | |- | ||
| + | | | ||
| + | ::We subtract 6 from 48; 42 remains and this is the amount of money he has. | ||
| + | ::<math>\scriptstyle{\color{blue}{48-6=42}}</math> | ||
| + | |style="text-align:right;"|נגרע מספר ו' ממ"ח וישאר מ"ב והם המעות שבידו | ||
| + | |- | ||
| + | | | ||
| + | :*Or: he spends 18 pešiṭim every day and at the end of five days he has nothing left. | ||
| + | |style="text-align:right;"|או שיוציא בכל יום י"ח פשי' ולסוף ה' ימים לא נשאר לו מאומה | ||
| + | |- | ||
| + | | | ||
| + | ::One half and one part of 16, which is 9 parts of 16, is the number that when doubled in a double ratio as many times as the number of days, which is 5, the result is 18. | ||
| + | |style="text-align:right;"|הנה המספר שבהכפלו על יחס הכפל פעמים כמספר הימים שהוא ה' יעלה י"ח הוא חצי וחלק אחד מי"ו שהן ט' חלקים מי"ו | ||
| + | |- | ||
| + | | | ||
| + | ::Because, when we double one half and one part of 16, the result is 1 and one-eighth. | ||
| + | ::<math>\scriptstyle{\color{blue}{2\sdot\left(\frac{1}{2}+\frac{1}{16}\right)=1+\frac{1}{8}}}</math> | ||
| + | |style="text-align:right;"|כי כשנכפול חצי וחלק אחד מי"ו יעלה א' ושמינית | ||
| + | |- | ||
| + | | | ||
| + | ::When we double 1 and one-eighth, the result is 2 and a quarter. | ||
| + | ::<math>\scriptstyle{\color{blue}{2\sdot\left(1+\frac{1}{8}\right)=2+\frac{1}{4}}}</math> | ||
| + | |style="text-align:right;"|וכשנכפול א' ושמינית יעלה ב' ורביע | ||
| + | |- | ||
| + | | | ||
| + | ::When we double 2 and a quarter, the result is 4 and a half. | ||
| + | ::<math>\scriptstyle{\color{blue}{2\sdot\left(2+\frac{1}{4}\right)=4+\frac{1}{2}}}</math> | ||
| + | |style="text-align:right;"|וכשנכפול ב' ורביע יעלה ד' וחצי | ||
| + | |- | ||
| + | | | ||
| + | ::When we double 4 and a half, the result is 9. | ||
| + | ::<math>\scriptstyle{\color{blue}{2\sdot\left(4+\frac{1}{2}\right)=9}}</math> | ||
| + | |style="text-align:right;"|וכשנכפול ד' וחצי יעלה ט‫' | ||
| + | |- | ||
| + | | | ||
| + | ::And when we double 9 for the fifth time, the result is 18. | ||
| + | ::<math>\scriptstyle{\color{blue}{2\sdot9=18}}</math> | ||
| + | |style="text-align:right;"|וכשנכפול חמשית ט' יעלה י"ח | ||
| + | |- | ||
| | | | ||
::We subtract a half and one part of 16 [from 18]; the result is 17 and 7 parts of 16 and this is the amount of money he has. | ::We subtract a half and one part of 16 [from 18]; the result is 17 and 7 parts of 16 and this is the amount of money he has. | ||
| Line 2,471: | Line 3,555: | ||
|style="text-align:right;"|נגרע מספר חצי וחלק אחד מי"ו יעלה י"ז וז' חלקים מי"ו והם המעות שבידו | |style="text-align:right;"|נגרע מספר חצי וחלק אחד מי"ו יעלה י"ז וז' חלקים מי"ו והם המעות שבידו | ||
|- | |- | ||
| − | | | + | |The reason the root is written in the middle rank, whether there is an odd number of ranks, or an even number, is that we shift the even rank above the preceding rank and add them together, as when we write 7 above the 9 and it becomes seventy. |
| − | |style="text-align:right;"|טעם למה יוציא השרש אל אמצע המדרגות בין שיהיו המדרגות נפרדים כמו זה או זוגות שנשים המדרגה הזוגיית למעלה מהמדרגה שלפניה שנחבר הכל כמו שנשים | + | |style="text-align:right;"|‫<ref>80r</ref>טעם למה יוציא השרש אל אמצע המדרגות בין שיהיו המדרגות נפרדים כמו זה או זוגות שנשים המדרגה הזוגיית למעלה מהמדרגה שלפניה שנחבר הכל כמו שנשים <sup>ז'</sup> למעלה מט' ויהיה שבעים |
| + | |- | ||
| + | |Since in every product, the ranks are doubled. After we square it, the product has six ranks. | ||
| + | |style="text-align:right;"|לפי שלעולם בכל כפל המדרגות כפולות אחר שנעשה מהם מרובע מהכאתם עולה לששה מדרגות | ||
| + | |- | ||
| + | |If the result of multiplication has an even [number of ranks]: | ||
| + | |style="text-align:right;"|אם יש ליוצא מההכאה זוגות | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :As here, where 8 by 8 is 64. So, 64 is beneath the sixth rank. |
| + | |style="text-align:right;"|כמו זה שח' על ח' עולה לס"ד ולכן ס"ד הוא תחת המדרגה הששית | ||
|- | |- | ||
| − | | | + | |If the number [of the ranks] is odd, we take the root from [...] |
|style="text-align:right;"|ואם המספר נפרד לא נקח שרש כי אם מה | |style="text-align:right;"|ואם המספר נפרד לא נקח שרש כי אם מה | ||
|- | |- | ||
|} | |} | ||
| + | |||
== Notes == | == Notes == | ||
| + | <div style="text-align: right;"><div class="mw-collapsible mw-collapsed"><div class="mw-collapsible-content"> | ||
| + | <br> | ||
| + | <references group=note/> | ||
| + | </div></div></div> | ||
| + | |||
| + | == Apparatus == | ||
| + | |||
| + | <div style="text-align: right;"><div class="mw-collapsible mw-collapsed"><div class="mw-collapsible-content"> | ||
| + | <br> | ||
<references /> | <references /> | ||
| + | </div></div></div> | ||
== Appendix: Bibliography == | == Appendix: Bibliography == | ||
Latest revision as of 18:46, 4 November 2024
Contents
- 1 Introduction - Decimal Fractions
- 2 Table – multiplication of sexagesimal fractions (Firenze)
- 3 Table – multiplication of sexagesimal fractions (Firenze)
- 4 Multiplication of integers (Firenze)
- 5 Division of integers
- 6 Extracting roots
- 7 Division of sexagesimal fractions
- 8 Extracting roots of sexagesimal fractions
- 9 Multiplication of sexagesimal fractions
- 10 A Rule for Checking the Squares
- 11 MS London 7r-v
- 11.1 Chapter on Summation
- 11.2 Word Problems
- 11.2.1 "How Many" Problem
- 11.2.2 "If You Give Me" Problem
- 11.2.3 "Find the Amount" Problem
- 11.2.4 Pursuit Problem
- 11.2.5 Encounter Problem
- 11.2.6 Payment Problem
- 11.2.7 Boiling Problems
- 11.2.8 Whole from Parts Problems - lance
- 11.2.9 Multiple Quantities Problems
- 11.2.10 Whole from Parts Problems - fish
- 12 Glosses on Abraham Ibn Ezra’s Book of the number (P1026)
- 12.1 The author of the Sefer Yezira
- 12.2 Sefar
- 12.3 Sefer
- 12.4 Sipur
- 12.5 Since
- 12.6 Higher decade
- 12.7 The remainder is the required
- 12.8 The sum is the required
- 12.9 Example: we wish to multiply 29 by 31
- 12.10 Know that if there are two digits to multiply
- 12.11 You have to
- 12.12 As if they are units
- 12.13 Does not assume any change
- 12.14 Does not assume any increase
- 12.15 Does not assume any division
- 12.16 It is the cause of any change
- 12.17 According to its rank
- 12.18 Less than the number
- 12.19 Did not reach the rank of the units
- 12.20 Not gone completely
- 12.21 We always subtract backwards
- 12.22 One is like a point
- 12.23 Consists of surfaces
- 12.24 It is similar to an odd number
- 12.25 It is a half of an eighth
- 12.26 But I shall teach you a short method
- 12.27 The difference between the fifth and the ninth is four
- 12.28 Example: The ratio of 4 to [6]
- 12.29 Its proportions are composed
- 12.30 Because the ratio is always
- 12.31 Question: Reuven hired Shimon
- 12.32 And you do not know the third: multiply the first by the second
- 13 Additional Word Problems (P1026)
- 14 Notes
- 15 Apparatus
- 16 Appendix: Bibliography
Introduction - Decimal Fractions |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The division method of R. Immanuel | [1]דרך חלוק לר' עמנואל וע'א' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Introduction | הקדמה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Know that the one is divided into ten parts called primes; each prime is divided into ten parts called seconds and so on endlessly. | דע כי האחד נחלק לעשרה חלקים יקראו ראשונים וכן כל ראשון נחלק לעשרה חלקים יקראו שניים וכן לאין תכלית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Similarly I want to remind you that I call the rank of tens of integers "primes"; the hundreds of integers "seconds" and so on endlessly. | וכן אמרתי להזכירך כי הנני קורא למעלת העשרות שלימים ראאשונים ולמאות שלמים שניים וכן לאין תכלית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| I call the rank of units by their name "units", since it is mean between the integers and the fractions, therefore, when the units are multiplied by units the result is units. | אמנם מעלת האחדים אני קורא אותם בשמם אחדים לפי שהוא אמצעי בין השלמים והשברים ולזה כשיכפל אחדים באחדים יצאו אחדים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| I call the ranks whose name is greater "having a greater name", meaning I call the thirds "having a greater name than" the seconds, since the [name of] the thirds is derived from three and [the name of] the seconds [is derived] from two; likewise the fourths "having a greater name than" the thirds, and so the fifths ["having a greater name than"] the fourths. This is for the integers as well as for the fractions. | וכן אני קורא המעלות שהם גדולות השם גדול השם רצוני בזה שלישיים אני קורא יותר גדול השם משניים לפני ששלישיים נגזר משלשה ושניים משנים וכן רביעיים יותר גדול השם משלישיים וכן חמשיים מרביעיים וזה הוא בשלמים ובשברים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| When I say: add the name of this to the name of this, or subtract the name of this from the name of this: | וכן כשאומר חבר שם זה עם שם זה או גרע שם זה משם זה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| I mean add the name of the seconds to the name of the thirds and they are fifths.
|
רצוני בזה חבר שם שניים עם שם שלישיים ויהיו חמשיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If the name of the seconds to the name of the seconds, they are fourths.
|
ואם שם שניים עם שם שניים יהיו רביעיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Also, subtract the name of the seconds from the name of the thirds; primes remain.
|
וכן גרע שם שניים משם שלישיים וישאר ראשונים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If the name of the seconds from the name of the seconds, nothing remains, so they are in the rank of the units.
|
ואם שם שניים משם שניים לא ישאר דבר ויפול במעלת האחדים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| This is for integers as well as for fractions. | וזה בשלמים ובשברים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| When you subtract a greater name from a smaller name, as if we say: we subtract fourths from seconds, whether fractions, or integers; the result is in the rank of seconds of the other type. | וכאשר תגרע שם גדול משם קטן כאמרנו נגרע רביעיים משניים הן בשברים [הן בשלמים][2] יביא במעלת השניים לצד האחר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| As if we say: we subtract the name of the fourths that are fractions from the name of the seconds that are fractions; the result is in the rank of seconds that are integers.
|
כאמרנו נגרע שם רביעיים בשברים משם שניים גם כן בשברים יפול במעלת שלמים שניים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| When we say this for integers, i.e. we wish to subtract the name of the fourths that are integers from the name of the seconds that are integers, the result is of the seconds that are fractions.
|
וכן באמרנו זה בשלמים ר"ל שנרצה לגרוע שם רביעיים שלמים משם שניים שלמים יפול בשברים שניים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| When you multiply a number by a number and they are both integers or both fractions: | כשתכפול מספר על מספר ושניהם שלמים או שניהם שברים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Add the names of the ranks [one to the other] and the product is [in the rank whose name is their sum], of the integers, if both are integers, or of the fractions, if both are fractions.
|
חבר שם המדרגות האחד ושם היא הנכפל בשלמים אם שניהם שלמים ובשברים אם שניהם שברים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If [one is] integer and the other fraction: | ואם שלמים והאחר שברים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If their names are the same, the product is in the rank of units.
|
אם הם שוים בשם הנה יכפול הנכפל במעלת האחדים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If the name of one is greater than the other, subtract the smaller from the greater and the product is as the name that remains: integers, if the [name of] the integers is greater, or fractions, if the [name of] the fractions is greater.
|
ואם שם האחד רב על האחר גרע הקטן מהגדול וכמספר השם שישאר שם יפול הנכפל בשלמים אם הם שלמים היותר גדול או בשברים אם שם השברים הוא יותר גדול | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| When you divide a number by a number and they are both integers or both fractions: | כשתחלק מספר על מספר ושניהם שלמים או שניהם שברים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If the names of their ranks are the same, the quotient is in the rank of units. Because, when you subtract this name from this name, nothing remains, so it is in the rank of units.
|
ושם מדרגותיהם שוה הנה יפול החלוקה במעלת האחדים לפי שכאשר תגרע שם זה משם זה לא ישאר דבר ויפול במעלת האחדים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If [the name of] the upper is greater than the name of the lower, subtract the name of the lower from the name of the upper and the quotient is as the name that remains of the same type, i.e. integers, if [both are] integers, or fractions, if [both are] fractions.
|
ואם העליון יותר גדול השם מתחתון גרע שם התחתון משם העליון וכמספר שֵם הנשאר יפול החלוקה בצד ההוא ר"ל בשלמים אם הי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If [the name of] the lower is greater, subtract the name of the greater from the name of the lower and the quotient is as the name that remains of the opposite type: fractions, if both are integers, or integers, if both are fractions.
|
ואם התחתון יותר גדול גרע שם העליון משם התחתון וכמספר שם הנשאר יפול החלוקה בהפך הצד ר"ל בשברים אם היו שניהם שלמים או בשלמים אם היו שניהם שברים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If one is integer and the other is fraction and the names of their ranks are the same, or not the same, add the names of their ranks and the quotient is as the name of the sum: fraction, if the upper is a fraction, or integer, if the upper is an integer.
|
ואם האחד שלמים והשני שברים ושם מדרגותיהם שוה או בלתי שוה חבר שם המדרגות וכמספר שם העולה בשברים אם העליון שברים או בשלמים אם העליון שלמים שם יפול החלוקה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Another Version - MS Paris 903 |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Know that the one is divided into ten parts called primes; each prime [is divided] into ten parts called seconds, each second into ten thirds, each third into ten fourths and so on endlessly. | דע כי האחד נחלק לי' חלקים יקראו שלמי'שברי' ראשוני' וכל אחד מהראשוני' לי' חלקי' יקראו שלמי' שברי' שניים וכל שניי' לי' שלישיי' וכל שלישיי' לי' רביעיי' וכן עד אין קץ | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| I call the rank of tens of integers "primes"; the hundreds of integers "seconds", the thousands of integers "thirds", the tens of thousands of integers "fourths", the [hundreds of thousands] "fifths" and so on endlessly. | וכן אני קורא מעלת העשרו' שלמים ראשונים ולמאות שלמי' שניי' ולאלפי' שלמי' שלשיי' ולרבואות שלמים רביעיי' ולרבבות חמשיי' וכן עד אין קץ | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| I call the rank of units by their name "units", since they are mean between the integers and the fractions, therefore, when the units are multiplied by units the result is units, which does not happen in any other rank. | אך האחדי' אני קור' בשמם אחדי' כי הם כאמצעיים בין השלמי' והשברי' על כן בכפל אחדי' באחדי' יצאו אחדי' מש"כ בשום מעל' אחרת | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| I call the seconds "having a greater name than" the primes, the thirds ["having a greater name than"] the seconds, and so on. This is for integers as well as for fractions. | וכן אני קורא לשניי' גדולי השם מראשוני' וכן שלישיים משניי' וכן כלם בי' בשלמי' בי' בשברי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| When I say: add the name of the seconds to the name of the thirds, the result is fifths and so on.
|
וכן באמ' חבר שם שניי' עם שם שלישיי' יצא חמשיי' וכן כלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Also, when I say: subtract the name of the primes from the name of the seconds; primes remain.
|
וכן באמ' גרע שם ראשוני' משם שניים ישאר ראשוני' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If from the name of the primes, nothing remains, so they are in the rank of the units, for integers as well as for fractions.
|
ואם משם ראשונים לא ישאר דבר ויכפול במעל' האחדי' בי' בשלמי' בי' בשברי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If the subtracted has a greater name than the minuend, as if you subtract fourths from seconds, if they are fractions, the result are seconds of integers, and if they are integers, the result are seconds of fractions.
|
ואם הנגרע גדול השם מאשר גרעון ממנו כגון שתגרע רביעיי' משניי' אם הם בשברי' יצאו שניי' שלמי' ואם הם בשלמי' יצאו שברי' שניים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| When you multiply integers by integers or fractions by fractions: | וכשתכפל שלמי' בשלמי' או שברי' בשברי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Add the names of the ranks [one to the other] and the product is [in the rank whose name is their sum].
|
חבר שם המדרגו' ושם יפול הנכפל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| When you multiply integers by fractions: | וכשתכפל שלמי' בשברי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If their names are the same, the product is in the rank of units.
|
אם הם שוים בשם יפול הנכפל באחדי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If not, subtract the names of the smaller from the name of the greater and the product is as the name that remains after the subtraction.
|
ואם לאו גרע שם הקטן מן שם הגדול ושם יפול הנכפל [..] בצד הגדול בשם הנשאר אחר הגרעון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| As if you multiply 3 seconds of integers by 2 sevenths of fractions: subtract 2 from 7; the result is 5. We find that the result of the multiplication is 6 fifths of fractions.
|
כאלו תכפול ג' שלמי' שניי' בב' שברי' שביעיי' תגרע ב' מז' וישאר ה נמצ' יעלה הכפל ו' שברי' חמשיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If 3 seconds of fractions by 2 sevenths of integers, the product is 6 fifths of integers.
|
ואם ג' שברי' שניי' בב' שלמי' שביעיי' יהיה הנכפל ו' שלמי' חמשיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| This is the rule: when you divide a number by a number and they are both integers or both fractions: | וזו לך צורה לזה כשתחלק מספר על מספר ושניהם שלמי' או שניה' שברי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If the names of their ranks are the same, the quotient is always in the rank of units.
|
אם שם מדרגותיה' שוה תפול החלוק באחדי' לעולם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If the name of the upper is greater than [the name of] the lower, subtract the name of the lower from [the name of the upper] and the quotient is as [the name] that remains [of the same type], i.e. integers, if [both are] integers, or fractions, if [both are] fractions.
|
ואם שם העליון גדול מהתחתון גרע שם התחתון ממנו וכמספר הנשאר תפול החלוקה בצד ההו' ר"ל אם הם שלמי' שלמי' ואם שברי' שברי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If the name of the lower is greater, subtract [the name of] the greater from [the name of the lower] and the quotient is as [the name] that remains of the opposite type i.e.: fractions, if both the divisor and the dividend are integers, or integers, if both are fractions.
|
ואם שם התחתון גדול גרע העליון ממנו וכמספר הנשאר תפול החלוק בהפך הצד ר"ל בשברי' אם החולק והנחלק שלמי' ובשלמי' אם הם שברים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If one is integer and the other is fraction and the names of their ranks are the same, or not the same, add the names of their ranks and the quotient is as the name of the sum, of the type of the upper number, i.e. the dividend: integer, if [the upper] is an integer, or fraction, if [the upper is] a fraction,.
|
ואם האחד שלם והשני שברי' יהיה שם מדרגותיהם שוה או בלתי שוה חבר שמות המדרגו' יחד וכמספר המחובר יהיה שם הנופל בחלוק לצד שבו היה המספר העליון ר"ל המתחלק אם שלמי' בשלמי' ושברי' בשברי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
MS Paris 1081 15r-16v |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Said Immanuel the son of Jacob: Since the division procedure is more difficult for the students than the multiplication procedure, and this is the way for the wise arithmeticians to extract the unknown number from the knowledge of the remaining five numbers and this is done by two multiplications and two divisions in different ways, we found it appropriate to explain how to extract the unknown from the knowledge of the remaining five and this is done by three multiplications and one division and I first give some signs for this procedure: | [3]אמר עמנואל בן יעקב לפי שמעשה החלוק יותר קשה למתלמדים ממעשה הכפל והיא הדרך לשאר החשבנים החכמים להוציא המספר האחד הנעלם איזה שיהיה מתוך ידיעת החמשה מספרים הנשארים ויעשה בזה שני כפלים ושני חלקים בדרכים שונים ראינו לבאר להוציא הנעלם מתוך ידיעת החמשה הנשארים ויעשה בזה שלשה כפלים וחלוק אחד ואומר ראשונה לתת קצת אותות אל זה המעשה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Let the ratio of A to B consist of the ratio of G to D and the ratio of H to W.
|
הנה יחס א' אל ב' מחובר מיחס ג' אל ד' ומיחס ה' אל ו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| It has already been explained in [V].23 of Euclid that the ratio of the product of G by H to the product of D by W consists of the ratio of side G to side D and the ratio of side H to side W.
|
וכבר התבאר מתמונת כ"ג מששי אקלידיס שיחס השטח ההווה מן ג' בה' אל השטח ההוה מן ד' בו' מחובר מיחס צלע ג' אל צלע ד' ומיחס צלע ה' אל צלע ו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| So, the ratio of A to B is the same as the ratio of the product of G by H to the product of D by W.
|
אם כן יחס [א]' אל ב' כיחס השטח ההווה מן ג' בה' אל השטח ההווה מן ד' בו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Let the product of G by H be the number Z and the product of D by W be the number T.
|
ויהיה שטח ג' בה' מספר ז' ושטח ד' בו' מספר ט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Hence, the ratio of A to B is the same as the ratio of Z to T.
|
אם כן יחס א' אל ב' כיחס ז' אל ט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| We multiply the extremes, which are A by T; let it be L.
|
אם כן נכפול הקצות שהם א' בט' ויהיה ל' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| We divide L by the mean, which is B; the result is Z, which is the other mean.
|
ונחלק ל' על האמצעי והוא ב' ויעלה הז' שהוא האמצעי האחר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Or, we divide L by the mean, which is Z; the result is B, which is the other mean.
|
או נחלק ל' על האמצעי והוא ז' ויעלה ב' והוא האמצע האחר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Or, we multiply the means, which are B by Z; it is L. We divide it by one extreme, which is T; the result is A, which is the other extreme.
|
או נכפול האמצעיים שהם ב' בז' ויהיה ל' ונחלק על הקצה [4]האחד והוא ט' ויעלה א' שהוא הקצה האחר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| This is clear. | וזה מבואר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Thus, we explained [the case] when the first, which is A, is unknown, or the second, which is B, is unknown. | ובזה ביארנו אם הראשון והוא א' נעלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| It is explained in the eighteen propositions in the Conic Section of Menelaus that when the ratio of the first, which is A, to B, which is the second, consists of the ratio of G, which is the third, to D, which is the fourth, and the ratio of H, which is the fifth, to W, which is the sixth, then also the ratio of the third, which is G, to the fourth, which is D, consists of the ratio of the first, which is A to the second, which is B, and the ratio of the sixth, which is W, to the fifth, which is H. This is the eleventh proposition in the mentioned book.
|
מבואר בתמונה החתוכית המיוחדת למילאוס בשמנה עשר פנים שכאשר היה יחס הראשון והוא א' אל ב' והוא השני מחובר מיחס ג' והוא השלישי אל ד' והוא הרביעי ומיחס ה' אל ו' והוא החמשי אל ו' והוא הששי הנה גם כן יהיה יחס השלישי והוא ג' אל הרביעי והוא ד' מחובר מיחס הראשון והוא א' אל השני והוא ב' ומיחס הששי והוא ו' אל החמשי והוא ה' וזהו בפעם האחת עשרה עשרה מהתמונה הנזכרת | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Therefore, we set the ratio as follows: third, first, fourth, second, first, third, second, fourth, sixth, fifth, fifth, sixth. | אם כן נעשה היחס כן שלישי ראשון רביעי שני ראשון שלישי שני רביעי ששי חמשי חמשי ששי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Thus, according to the method we explained first, we can know [them] when the third is unknown, or when the fourth is unknown. | אם כן על הדרך שביארנו ראשונה נוכל לדעת אם ג' שהיה שלישי נעלם או אם שהיה רביעי נעלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| It is also explained in the seventeenth proposition in the mentioned book that when the ratio of A, which is the first, to B, which is the second, consists of the ratio of G, which is the third, to D, which is the fourth, and the ratio of H, which is the fifth, to W, which is the sixth, then also the ratio of the fifth, which is H, to the sixth, which is W, consists of the ratio of the first, which is A to the second, which is B, and the ratio of the fourth, which is D, to the third, which is G.
|
וגם כן יתבאר בפעם השבעה עשר מהתמונה הנזכרת שכאשר יחס א' והוא הראשון אל ב' והוא השני מחובר מיחס ג' והוא השלישי אל ד' והוא רביעיי ומיחס ה' והוא החמישי אל ו' והוא הששי הנה גם כן יהיה יחס החמשי והוא ה' אל הששי והוא ו' מחובר מיחס הראשון והוא א' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Therefore, we set the ratio as follows: fifth, first, sixth, second, first, third, second, fourth, fifth, third, sixth. | אם כן נעשה היחס כן חמשי ראשון ששי שני ראשון שלישי שני רביעי חמשי שלישי ששי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Thus, according to the method we explained first, we can know [them] when H, which is the fifth, is unknown, or when W, which is the sixth, is unknown. | אם כן על הדרך שביארנו ראשונה נוכל לדעת אם ה' שהיה חמשי נעלם או ו' שהוא ששי נעלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The educated will understand. | והמשכיל יבין | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If the first, which is A, is unknown: we multiply G by H; it is Z. We also multiply B by W; it is T. We also multiply B by Z; it is L. We divide L by T; the result is A, which is the unknown first.
|
אם הראשון והוא א' נעלם נכה ג' בה' והיה ז' עוד נכה ב' בו' והיה ט' עוד נכה ב' בז' והיה ל' ונחלק ל' על ט' ויצא א' שהוא הראשון הנעלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If the [second], which is B, is unknown: we multiply G by H; it is Z. We also multiply B by W; it is T. We also multiply A by T; it is L. We divide L by Z; the result is B, which is the unknown second.
|
אם הששי והוא ב' נעלם נכה ג' בה' והיה ז' עוד נכה ב' בו' והיה ט' עוד נכה א' בט' והיה ל' נחלק ל' על ז' ויצא ב' שהוא השני הנעלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If the third, which is G, is unknown: we multiply A by W; it is Z. We also multiply B by H; it is T. We also multiply D by Z; it is L. We divide L by T; the result is G, which is the unknown third.
|
אם השלישי והוא ג' נעלם נכה א' בו' והיה ז' עוד נכה ב' בה' והיה ט' עוד נכה ד' בז' והיה ל' נחלק ל' על ט' ויצא ג' שהוא השלישי הנעלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If the forth, which is D, is unknown: we multiply A by W; it is Z. We also multiply B by H; it is T. We also multiply G by T; it is L. We divide L by Z; the result is D, which is the unknown fourth.
|
אם הרביעי והוא ד' נעלם נכה א' בו' והיה ז' עוד נכה ב' בה' והיה ט' עוד הכה ג' בט' והיה ל' נחלק ל' על ז' ויצא ד' שהוא הרביעי הנעלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If the fifth, which is H, is unknown: we multiply A by D; it is Z. We also multiply B by G; it is T. We also multiply W by Z; it is L. We divide L by T; the result is H, which is the unknown fifth.
|
אם החמשי והוא ה' נעלם נכה א' בד' והיה ז' עוד נכה ב' בג' והיה ט' עוד נכה ו' בז' והיה ל' נחלק ל' על ט' ויצא ה' שהוא החמשי הנעלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If the sixth, which is W, is unknown: we multiply A by D; it is Z. We also multiply B by G; it is T. We also multiply H by T; it is L. We divide L by Z; the result is W, which is the unknown sixth.
|
אם הששי והוא ו' נעלם נכה א' בד' והיה ז' עוד נכה ב' בג' והיה ט' עוד נכה ה' בט' והיה ל' נחלק ל' על ז' ויצא ו' שהוא הששי הנעלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| This is about the way I explained to extract the unknown from the six by the knowledge of the remaining five. Understand [this]. | זהו על דרך שבארתי להוציא הנעלם איזה שיהיה מהששה מתוך ידיעת החמשה הנשארים ובין | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Table – multiplication of sexagesimal fractions (Firenze) |
לוח כפילת המעלות ושבריהם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Table – multiplication of sexagesimal fractions (Firenze) |
לוח חילוק השברים האחד על השני | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Multiplication of integers (Firenze) |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון על דרכי הכפל רצינו לכפול קכ"ז על שנ"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכתבנו בטור העליון קכ"ז ובשני השפל שנ"ה וזה צורתו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפלנו ז' על ה' והוא ל"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כתבנו ה' במעלה הראשונה כנגד ז' ושמרנו הג' כדי לצרף אותה אל המעלה השנייה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד כפלנו ז' על ה' השני התחתון עלו ל"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חברנו הה' עם הג' ששמרנו ועלו ח' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ושמנו ח' במעלה השנית תחת ב' מטור העליון והג' שמנו במעלה השלישית תחת הא' מטור העליון עם היוצא מכפל אותה המעלה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד כפלנו ז' הראשון על הג' התחתון עלו כ"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חברנו א' עם הג' ששמרנו והיו ד' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכתבנו ד' תחת הא' במעלה השלישית וב' במעלה רביעית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד כפלנו ב' האמצעי העליון על ה' הראשון מן הטור השפל עלו עשר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כתבנו ציפרא בשינית וא' בשלישית תחת הג' שבטור השפל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד כפלנו ב' העליון על הה' השני התחתון עלו עשר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חברנו י' עם הא' ששמרנו והיא י"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שמנו א' בשלישית וא' שמרנו ברביעית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד כפלנו ב' על ג' והיו ששה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חברנו הו' עם הא' והיו ז' וכתבנוהו ברביעית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד כפלנו א' על ה' הראשונ שבטור השפל וכתבנו ה' בשלישית עוד כפלנו א' על ה' הראשון שבטור השפל וכתבנו ה' בשלישית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד כפלנו א' על ה' השני וכתבנו ה' ברביעית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד כפלנו א' על ג' וכתבנו ג' במעלה החמישית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נשלם כפל החבור | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נבוא לחבר היוצא מכפל שני הטורים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונכתוב ה' לכח במעלה הראשונה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וח' בשנית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וד' וא' וה' עולים עשרה נעשה 0' בשלישית ונשים נקודה ברביעית להיות לזיכר בעבו' העשרה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואחר נחבר ב'ז'ה' והם י"ד ואחר השמור הרי ט"ו נשים ה' במעלה הרביעית ונקודה בחמישית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נחבר אחד עם הג' ויהיו ד' ונכתוב ד' במעלה החמשית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נשלם החבור | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The scales of multiplication by [casting out] the nines | המאזניים מן הכפל על חש' ט' ט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה חשבנו חשבון הטור העליון כאילו הם אחדים והיו עשרה כזה הדרך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
השלכנו התשעה ונשאר א' וכתבנוהו בצד הטור העליון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אחר כן חשבנו הטור השפל ומצאנוהו עולה י"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הפלנו הט' ונשארו ד' וכתבנום בצדו אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואחר כפלנו א' על ד' והיה ד' ושמרנום | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואחר חשבנו המחובר והיה זה ה ח 0 ה ד ועלה כ"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
השלכנו י"ח שהולך לתשיעיות ונשארו ד' והוא שווה לכפל מאזני שני הטורים ואז ידענו שחשבונינו אמתי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The rule is that when one of the multiplicands of the product is cast out by nines, whether the upper or the bottom, then the result of the multiplication should be cast out by nines; otherwise not. | והכלל הוא כי כשאחד ממדרגות הכפל הולך לתשיעיות הן העליון הן השפל היוצא מחבור הכפל ראוי ללכת בתשיעיות ואם לאו אינו צורך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If both are not removed by nines, multiply the remainders of the two rows of multiplication by each other and see the remainder from nines, whether it is 5, or 6, or 7. | ואם אינו הולך לתשיעיות כל אחד משניהם תכפול העודף משני טורי הכפל זה על זה וראה מה שיעדיף על תשיעיות אם ה' אם ו' אם ז' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| That is, see the result of the upper row and multiply it by the result of the bottom row. | ר"ל תראה מה שיעלה הטור העליון ותכפלהו על מה שיעלה הטור השפל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כאלו יהיה בעליון ד' ג' שעולה ז' ובתחתון ג' ב' שעולה ה' תכפול ז' על ה' עולה ל"ה נשאר על תשיעיות שהשלכת ח' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The same will be in the product no more and no less, when you examine how much is the result of the multiplication and by how much it exceeds over nine. | וכן יהיה בחבור בלי פחות ויתר כאשר תעיין כמה חבור הכפל וכמה יעדיף על תשעה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If the total of the upper row and of the bottom row are less than 9, multiply the upper by the bottom, see by how much [the product] exceeds over nines and the same should be for the result of the multiplication, if the multiplication is correct; otherwise, it is incorrect. | ואם בין הטור העליון והשפל לא יעלה הכל ט' תפיל העליון על השפל וראה מה שיעדיף על תשיעיות וככה יהיה החבור אם הכפל יצדק ואם לא יצדק תם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Division of integers |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Conducted by Bonfils may his soul rest in paradise. | [6]עשאו שין בונפייל נ"ע[7] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| When you want to divide many digits by many digits, however many there may be, write the number you wish to divide, which is the greater, in one row, each one according to its rank and call it "the upper row". | כשתרצה לחלק מספרים רבים על מספרים רבים כמה שיהיו תשים המספר שתרצה לחלקו והוא ביותר גדול בטור כל אחד ואחד כפי מעלתו וקרא טור עליון[8] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Write the second number, by which you want to divide, and it is the smaller, in another row, beneath the upper row, each beneath its type, i.e.: units beneath units, tens beneath tens etc., and this row is called "the bottom row". | והמספר השני שתרצה לחלק עליו והוא היותר קטן תשים בטור אחר תחת הטור העליון כל מין תחת מינו ר"ל אחדים תחת אחדים עשרות תחת עשרות וכו' שהטור יקרא הטור השפל[9] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Leave a space between the two rows we mentioned, so that you can write the result of division between them and this row is called "the middle row". | וריוח תשים בין שני הטורים שהזכרנו כדי שתוכל לכתוב ביניהם העולה בחלוק וזה הטור יקרא טור אמצעי[10] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| When you start dividing your number, consider all the digits as if they were units and see how many times the last digit of the bottom row is in the last digit of the upper row. | וכאשר תחל לחלק חשבונך ותחשוב כל המספרי' כאלו הם אחדים תראה כמה פעמים יהיה המספר האחרון שבטור השפל כמספר אחרון שבטור עליון[11] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| In such way that the second to the last in the bottom row, i.e. before it, is the same number of times in the second to the last in the upper row before it. | ובאופן שיהיה מספר הפעמים ההם השני לאחרון שבטור השפל ר"ל לפניו השני לאחרון שבטור העליון לפניו[12] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Also the third to the last in the bottom row is in the third to the last in the upper row and so on successively. | וכן השלשי לאחרון שבטור | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Write the number you receive with the previous method, i.e. the number of times the last digit in the bottom row is in the last digit in the upper row, in the middle row, i.e. between the upper row and the bottom row. Write this number as far from the last digit of the upper row, from which you take the quotient, as the [number of] ranks that the last digit of the bottom row is far from the rank of units. | ואותו המספר אשר יעלה לך עם האופן הקודם ר"ל מספר הפעמים אשר יהיה המספר האחרון שבטור השפל כמספר האחרון שבטור העליון תכתוב בטור האמצעי ר"ל בין הטור העליון והשפל ותכתוב כמספר ההוא רחוק מהמספר האחרון שבטור העליון אשר תקח ממנו החלק כמו מדרגות אשר רחק כמספר האחרון שבטור השפל ממדרגת האחדים[14] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Know that if you cannot take the last digit of the bottom row even once from the last digit of the upper row, as if we say for instance that the last of the bottom row is 1 and the last of the upper row is 8 or less, like our next example: | ודע לך שאם לא תוכל לקחת שום פעם המספר האחרון שבטור השפל כמספר האחרון שבטור העליון כאלו נאמר דרך משל שהאחרון שבטור השפל הוא א' והאחרון שבטור העליון הוא ח' או פחות כמו בדמיונו זה[15] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אז תשוב כל המספר ההוא אחורנית ר"ל מספר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
לזה תקח ט' ותכתבנה א' בטור האמצעי רחוק מהאות שלקחת החלוק ממנו שהוא ט' בכמו המספר אשר רחק האות האחרון שבטור השפל שהוא ט' ממדרגתו והם ארבע מדרגות לכן תכתוב בט' אשר יצא לך בחלוק במדרגת העשרות שהוא תחת הב' שבטור העליון[17] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Then, draw a line beneath the bottom row and multiply the digit you received in the division, i.e. 9 by the whole bottom row, according to the multiplication method you know. Subtract the product from the upper row, each from its type, starting from the rank nearest to the units, or from the units, if there are units. Write the remainder from subtraction above the upper row. | אחר כן תשים קו תחת הטור השפל וכפול זאת האות שיצא לך בחלוק ר"ל ט' עם כל הטור השפל כאשר ידעת דרך הכפל והיוצא מהכפל גרע מהטור העליון כל מין ממינו ונתחיל מן המדרגה הקרובה אל האחדים או מן האחדים אם יש שם אחדים והנשאר אחר הגרעון[18] כתוב על הטור | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| After that, divide again what remains above the upper row by the bottom row in the manner we explained, then multiply the digit received from the division by the entire bottom row, as at the beginning, and subtract the product from what remains on the upper row and the remainder remains above it. | אחר זה שוב לחלק הנשאר על הטור העליון על הטור השפל על הדרך שביארנו ואחר כפול האות היוצא בחלוק על כל הטור השפל כאשר בתחלה ואחר גרע העולה מהכפל מן הנשאר בטור העליון וישאר הנשאר עליו[20] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Divide it again, if there is something in it to divide, multiply and subtract as mentioned. | ואחר תשוב לחלק עוד אם יש בה לחלק ולכפול ולגרוע כדבר האמור[21] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The sign for this method is D.M.S. [in Hebrew ḥ.k.m.], i.e. division, multiplication, and subtraction. | והסימן לזה הדרך ח'כ'ם' ר"ל חלוק כפל ומגרעת[22] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Always do this until what remains in the upper row is less than what is in the bottom row. | וכן תעשה תמיד עד שישאר בטור[23] עליון פחות ממה שבטור[24] השפל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| After you have done this and less remains in the upper row than in the bottom row and you want to check if your calculation was performed without making any mistakes, add up all the multiplication rows you received, each with its type and also what remains in the upper row and perform the addition operation, the procedure of which you are familiar with. If the sum is equal to the number you first had in the upper row, the calculation is correct, and if you find any change between them, there is an error. | וכאשר עשית זה ונשאר בטור העליון פחות ממה שבטור השפל ותרצה לבחון אם נעשית מלאכתך בזולת נפילת בה שום טעות תחבר כל טורי הכפל אשר יצאו לך כל מין עם מינו וגם הנשאר לך בטור עליון ותנהוג מנהג החבור אשר ידעת מנהגו ואם המחובר יהיה שוה למספר שיהיה לך ראשונה בטור העליון הנה החשבון אמיתי ואם תמצא שום התחלפות ביניהם הנה טעות ודוק ותשכח[25] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Table in MS Firenze 
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
MS Firenze margin:
|
שלא מן המאמ' והוא תוס' ביאור | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If you want to further divide the remainder from division in the procedure we did, decompose the remainder into the preceding rank. | ואם תרצה לחלק עוד הנשאר לחלק בזה הדרך שעשינו שבר הנשאר למעלה ולמדרגה שלפניה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| I.e. if the remainder are minutes, decompose them into seconds, or into thirds if the former were seconds, or to fourths, if they were thirds, and so on as long as the calculation continues. | ר"ל אם הנשאר הם מותר מדקים שברם לשניים וכן לשלישיים אם הקודמי' היו שניים וכן לרביעיים אם קדמו השלישיים וכן עד כולם כל אשר יימשך החשבון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Look at the previous division table and you will find the explanation of what the results are. | ועיין בלוח הקודם מהחילוק ותמצא ביאור העולים מה יהיו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Extracting roots |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| After I have explained to you the method of division in the easiest possible way, in addition to mentioning most of the number operations such as division, multiplication and subtraction, as well as addition and checking, I intend to clarify and inform an easy way to find the square roots of the numbers that have a real root, or the closest to the numbers that do not have a real root, whether they are integers alone, integers with fractions, or fractions alone; their rules and approximations, while mentioning the other methods of calculation also. | [26]אחר שביארתי לך דרך החלוק על הדרך היותר קל שאיפשר מצורף מה שיש בו מן ההזכרה מרוב דרכי המספר כמו חלוק כפל ומגרעת גם החיבור בבחינה אמרתי לבאר ולהודיע דרך קל למצא שרשי המרובעים מהמספרים שיש להם שורש אמיתי או היותר קרוב להם מהמספרים אשר אין להם שורש אמיתי שלמי' לבדם הן מהמספרים שיש שלמים עם נשברים או מנשברים לבדם הן וכלליהם ודקדוקהם ויהיה גם זה מן ההזכרה בשאר דרכי החשבון[27] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| From now on I will start and say: | ומעתה אתחיל ואומר[28] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Know that in the rank of units there are three square numbers, i.e. whose roots are known integers and they are: one, whose root is one; four, whose root is two; and nine, whose root is three.
|
דע כי במעלת האחדים יש בה שלשה מספרים מרובעים ר"ל ששרשיהם שלמים וידועים והם אחד ששרשו אחד וארבעה ששרשו שנים ותשעה ששרשו שלשה[29] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The second rank, which is the rank of tens, does not have a square number at all, only together with units, because ten is not a square number, nor twenty, nor 30, nor 40, nor 50, 60, 70, 80, 90. Yet with the addition of units, it has six squares, i.e. we add to the number of tens some units: like 16, whose root is 4; 25, whose root is 5; 36, whose root is 6; 49, whose root is 7; 64 whose root is 8; 81, whose root is 9. | ובמעלה השנית שהיא מעלת עשרות אין בה מספר מרובע כלל רק עם חבור אחדים כי עשרה אינו מספר מרובע ולא עשרים ולא ל' ולא מ' ולא נ' ס' ע' פ' צ' אמנם עם חבור אחדים יש בה ששה מרובעים ר"ל שנוסיף על מספר העשרות אחדים מה כמו י"ו שרשו ד' כ"ה שרשו ה' ל"ו שרשו ו' מ"ט שרשו ז' ס"ד שרשו ח' פ"א שרשו ט'[30] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Every rank of a number, whose numerical position is odd, such as third, fifth, seventh, ninth, and the like endlessly, is analogous to the rank of units. | וכל מעלת המספר שמספר מדרגתה נפרד כמו שלישית חמישית שביעית תשיעית ודומיהן לאין תכלית דומה למעלה האחדים[31] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| As, if you say, for example, the rank of hundreds, which is the third rank, also has three squares: | כאלו תאמר דרך משל במעלת המאיות שהיא מדרגת שלישית יש בה גם כן שלשה מרובעים[32] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והוא מאה שדומה לאחד שרשו עשרה שדומה לאחד[33] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וארבע מאות שדומה לארבע אחדים שרשו עשרים שדומה לשנים[34] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וט' מאות שדומה לט' אחדים שרשו שלשים שדומה לשלש[35] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Likewise every rank whose numerical position is even, like fourth, sixth, eighth, tenth, and so on endlessly, which is called an even rank, is analogous to the rank of tens, which has six squares by adding units to it as we have explained. So, the rank of thousands has six squares by adding hundreds, i.e. we shift each thousand backward as ten hundreds and add them to what is in the hundreds: | וכן כל מעלה שמספר מדרגתה זוג כמו רביעית ששית שמינית עשירית וכן לאין תכלית והיא אשר תקרא מעלה זוגיית דומה למעלה העשרות שיש בה ששה מרובעים עם חבור אחדים בה כמו שבארנו כן במעלת האלפים יש בה ששה מרובעים עם חבור המאיות ר"ל שנשים כל אלף ואלף אחורנית לעשרה מאיות ותחבר עם מה שנמצא במאיות[36] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כמו אלף ושש מאות הדומה לשש [37]עשרה שרשו ארבעים הדומה לארבעה[38] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואלפים ות"ק הדומה לכ"ה שרשו חמשים הדומה לחמשה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ושלשת אלפים ושש מאות הדומה אל ל"ו שרשו ששים הדומה לששה[39] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וארבעה אלפים ותשע מאות שדומה למ"ט שרשו שבעים שדומה לשבעה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וששת אלפים ארבע מאות הדומה לס"ד שרשו שמנים הדומה לשמנה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ושמנה אלפים ומאה הדומה לפ"א שרשו תשעים הדומה לתשעה[40] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| And so on every rank whose numerical position is even, like fourth, sixth, eighth, to infinity. | וכן כל מעלה שמספר מדרגתה זוג כמו רביעית ששית שמינית לאין תכלית[41] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| You should know that the result of any number falls in the middle rank of the ranks of that number. | ולך לדעת כי היוצא מכלל איזה מספר שיהיה יפול במעלת האמצעית שבמספר המדרגות[42] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כאלו תאמר דרך משל יש לנו מספר ד' במעלה השביעית שהוא מעלה נפרדת כמו הענין בזאת הצורה[43] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ושרשו ב' כמו שביארנו שראוי לכתוב ב' במעלה הרביעית שהיא מעלה האמצעית שיש לפניה ג' מדרגות וכהנה לאחריה[44] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Understand it for the other odd ranks. | ובין תבין בשאר המעלות הנפרדות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The same for the even rank: as we explained, there is no square in it, unless we return the last digit in the even rank backward, so each unit becomes a ten and we add everything to what is in the preceding rank. Then, the root should be extracted. The number of the ranks becomes odd, and every odd rank has a middle rank. | וגם במעלה הזוגיית כמו שבארנו אין בה שום מרובע אם לא בהשיב כל אחד מהמספר האחרון שבמעלה הזוגיית אחורנית ולעשות מכל אחד עשרה ולחבר הכל עם מה שבמעלה שלפניה ואז ראוי לקחת השרש ואז ישובו מספרי המעלות נפרד וכל נפרד יש לה מעלה אמצעית[45] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The resulting root should be written in the middle rank, i.e. as far from the last rank, from which it is extracted, as its distant from the units, because the units stand by themselves, in their rank and the root of the units is in the rank of the units. | וראוי לכתוב השרש היוצא במדרגת האמצעית ר"ל רחוק ממדרגה האחרונה אשר ילקח מהם כאשר מרחקו מן האחדים מפני שהאחדים עומדים בעצמם ובמקומם והוא שרש האחדים בטור האחדים[46] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The meaning is that the root is as far from the rank from which it is extracted as it is far from the units, i.e. the distance from the units is the same. | והענין אחר שהשרש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| After I have introduced this to you, I remind you the arrangement of the squares that are in the rank of units, which is the first: 1, 4, 9, whose roots are: 1, 2, 3 and in the rank of tens there are six squares: 16, 25, 36, 49, 64, 81 and their roots are: 4, 5, 6, 7, 8, 9. This is already known to you. | ואחר שהקדמתי לך זה אזהירך להזכירך מסורת המרובעים אשר במדרגת האחדים שהיא הראשונה שהם א' ד' ט' ושרשם א' ב' ג' ובמדרגות העשרות יש ששה מרובעים והם י"ו כ"ה ל"ו מ"ט ס"ד פ"א ושרשם ד' ה' ו' ז' ח' ט' ויהיה זה ידוע אצלך[48] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| I have already informed you that the odd rank follows the rule of the units and the even [rank] follows the rule of the tens. | וכבר הודעתיך כי המעלה הנפרדת הנה דינה כדין האחדים והזוגית דינה כדין העשרות[49] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Now I will teach you the procedure you apply in order to find the root of any number you want, whether it has a real root or the closest to it, if it does not have a real root: | ועתה אורה אותך דרך זו תלך בידיעת שרש איזה מספר שתרצה אם יש להם שרש [אמתי] או היותר קרוב לפניו אם אין להם שרש אמיתי[50] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Write the number, whose root you want to know, in one row by its ranks as many as they may be. | הנה תכתוב המספר אשר תרצה לדעת שרשו בטור אחד איזו מדרגות שתהיינה[51] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותאמר תחלה שתהיינה ה' מדרגות דרך משל א'ב'ג'ד'ה'[52] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה המעלה האחרונה היא נפרדת ודינה כדין האחדים לכן נדמה מספר ה' האחרון כאלו הוא ה' אחדים הנה שרשו היותר קרוב לו לפניו הוא ב' שהוא שרש ד'[53] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
לכן נכתוב ב' באמצע שהוא תחת אות הג' ונרשום קו תחת הב' עם מעט ריוח כדי שנוכל לכפול אות השרש היוצאת[54] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונכפול אות הב' שהוא השרש בעצמו והנה ראו[י] לכתוב הכפולה רחוק ממנה אחריה כאשר היא רחוקה מן האחדים וראוי לכתוב גלגלים כמספר המדרגות אשר לפניה והתחלתם כנגד הב' ביושר ובמדרגתה כאשר נאמר עתה כנגד הב' נתחיל [55]לכתוב ב' גלגלים כאשר אות הב' רחוקה מן האחדים שני מדרגות ואחר השני גלגלים נכפול הב' בעצמה ויהיה ד' ובין תבין בכל התחלת הכפולה וזכור לך זה ואחר גרע העולה מהכפל מהמספר הראשון מן במינו כאשר נודע המגרעת והנשאר ישאר על הטור העליון[56] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אחר תכפול השרש בשנים ר"ל שאם היה ג' תכתוב תחתיה ו' ואם ד' ח' ובדמיונו זה שהיה ב' תכתוב ד' והוא השרש הכפול בשנים[57] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תחלק הנשאר מהמספר הראשון על זאת הד' ותקח כל מה שתוכל באופן שתוכל לקחת מרובע המספר אשר יצא מהחלוקה כאלו תאמר בדמיונו זה הנה נשוב הא' אחורנית כי לא נוכל לקחת ד' ממנו אפי' פעם אחד ונשיבהו אחורנית עם הד' אשר לפניה ויהיה י"ד ונאמר כמה פעמים נוכל לקחת ד' מן י"ד הנה נקח ג' כי מהנשאר לפניו נוכל לקחת ג' פעמים ג' שהוא מרובע המספר היוצא מחלוקה ונכתוב ג' לפני השרש הראשון[58] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונכפול זאת הג על עצמה ראשונה ואחר על השרש הכפול[59] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונתחיל לכתוב זאת האות הנופלת א' בצדה ותעשה ראשונה גלגלים כאשר אות הג' רחוקה מהאחדים והוא גלגל אחד ראשונה כנגד הג'[60] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואחר נאמר ג' פעמים ג' הם ט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואחר נאמר ג' פעמים ד' הם ב'א' ותכתוב ב'א' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואחר נגרע זה הטור הנכפל מן הנשאר למעלה מן במינו כמשפט המגרעת והנשאר ישאר למעלה בטור העליון[61] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אחר זאת תכפול זאת האות השנית מן השרש שהיא ג' בשנים ונכתוב ו' תחתיה[62] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונקח כל מה שנוכל באופן שנוכל לקחת באחרונה מרובע המספר היוצא מן החלוקה כאלו תאמר בדמיונו זה הנה נשוב הא' אחורנית כי לא נוכל לקחת ד' ממנו ויהיו י"ד ונקח ג"א כי מהנשאר נוכל לקחת ג' פעמים ומהנשאר אחר זה נוכל לקחת ג' פעמים ג' ונכתוב ג' לפני הו' והיא במעלת האחדים[63] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונכפול זאת הג' ראשונה בעצמה והוא ט' ונכתבנה כנגדה לפי שאות הג' היא במעלת האחדים[64] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אחר נכפול ג' על ו' ואחר ג' על ד' ואחר נגרע העולה מהכפל מן הנשאר למעלה והנשאר ישאר למעלה[65] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וישאר למעלה ל"ב והשרש ג'ג'ב' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונשלם באורו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| I will give you various rules, the knowledge of which is necessary for the root extraction procedure. | אך אמנם אתן לך כללים מתחלפים יש צורך בידיעתם במעשה השרשים[66] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הכלל הראשון כאשר לקחת השרש הראשון מהמספר האחרון אשר בידך אם היא מדרגת נפרדת או מהקודם לאחרון אם הוא מדרגת זוגית ראוי לכתוב השרש ההוא במדרגת האמצעית כאשר הראיתיך ותקח כל מה שתוכל ואם לא תוכל לקחת דבר מהמקום ההוא האחרון הנשאר ולקחת מהשני לו גם כן תכתבהו רחוק מהמקום אשר תקח ממנו החלוק כאשר השרש הראשון רחוק מהאחדים ולכן ראוי לכתוב גלגל במקום אשר לפני השרש הראשון וקודם [67]לזה הגלגל תכתוב היוצא מן החלוקה[68] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הכלל השני כאשר תשוב לחלק הנשאר מהמספר אשר בידך כפול השרש לא תקח המספר האחרון אשר לקחת ממנו ראשונה ואף כי מהמספר אשר אחריו ואף כי תראה כי האפשר לקחת בו מפני שכאשר לוקח ממנו השרש ראשונה כבר לוקח ממנו כל הראוי כמו שנראה בדמיונו זה[69] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
רצינו לדעת השרש הקרוב אל ט'ט'ג'[70] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה לקחנו השרש הקרוב והוא א' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וגרענו א' מהמספר האחרון שהוא ג' ונשאר ב' על הג'[71] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונכפול אות הא' והיא הב'[72] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולא נאמר ולראות כמה פעמים יהיה ב' שהוא כפל השרש במספר הנשאר ממספר הראשון והוא ב' מאשר נוכל לקחת פעם אחת ב' מב' וגם נוכל לקחת מרובע א' מהנשאר זה אין ראוי לעשות מפני כי יהיה מהראוי לכתוב זה הא' היוצא רחוק מהמספר אשר לקחנו ממנו החלוק כאשר אות הא' הנכתב ראשונה נכתב כמשפט הזה וכבר לקחנו כל המצטרך במדריגה ההיא ולכן נשוב הב' אחורנית על הט' אשר לפניה ויהיה כ"ט[73] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונאמר כמה פעמים יהיה ב' שהוא כפל השרש במספר כ"ט באופן שנוכל לקחת מהמדרגה שלפניה מרובע המספר היוצא מהחלוקה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ודי בזה זה הכלל והוא נמשך עם הכלל השלישי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הכלל השלישי כאשר שבת לחלק המספר הנשאר על השרש הכפול לא תטריח בעצמך לראות אם תוכל לקחת עשרה פעמים המחלק במחולק כמו שנאמר בדמיונו שלמעלה מזה נראה כמה פעמים יהיה ב' במספר כ"ט ונאמר שיהיה בו עשרה כי זה אי אפשר ואף כי יותר מעשרה כי לא תוכל לקחת מהמדרגה שלפנינו עשרה פעמים עשרה ואין ראוי לטרוח בזה וכן זה הדין בענין החלוק[74] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הכלל הרביעי מאשר אמרנו בכלל השני כי כאשר נשוב לחלק הנשאר על [כפל] השרש שלא נשוב לקחת מהמדרגה אשר לקחנו ראשונה זהו כאשר היה כשכפל[נו] זה השרש לא הוספנו עליו מדרגה כמו אם כפלנו ב' היה ד' באותה מדרגה וכן ג' וכן ד' אמנם אם היה השורש ה' או ו' או ז' או ח' או ט' והנה כשכפלנו זה השורש כבר נתוספה בשורש מדרגה אחת כאלו תאמר היה השורש ו' עשרות הנה כפל השורש ב' עשרות ומאה הנה ותוספת השרש מדרגת אחת ולפי זה כאשר תשוב לחלק תקח מהמדרגה אשר לקחת ממנו ראשונה ותכתבנה גם כן רחוקה מהמספר האחרון כאשר ראש השרש הכפול רחוק מן האחדים וזהו מה שרצינו לבאר[75] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Another Version - MS Paris 903 |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The way to extract a square root of a number that has a real root, or an approximate square root of those that do not have a real root, for integers alone, or for fractions alone, or for integers and fractions together. | [76]הדרך להוציא שרשי המרובעי' ממספר בעל שרש אמתי או שרש המרוב' הקרוב מאשר אי' להן שרש אמתי
הן משלמי' לבד או נשברי' לבד הן ממספר שלמי' ונשברי' יחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Know that in the rank of units there are three square numbers, i.e. their roots are integers and are known, and they are 1, 4, 9, whose roots are 1, 2, 3.
|
דע כי במעלת האחדי' יש בה ג' מספרי' מרובעי' ר"ל ששרשם שלמי' וידועי' והם א' וד' וט' ששרשם א' ב' ג' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The second rank, which is [the rank of] tens, does not have a square number at all, only together with units, because 10, 20, 30, 40, 50, 60, 70, 80, 90 none of them is a square number. Yet with the addition of units, it has six squares: like 16, whose root is 4; 25, whose root is 5; 36, whose root is 6; 49, whose root is 7; 64 whose root is 8; 81, whose root is 9. | ובמעלה השנית שהי' עשרות [אין בה] מספר מרובע כלל רק עם חבור אחדי' כי י'כ'ל'מ'נ'ס'ע'פ'צ' אי גם אחד מהם מרובע אך עם חבור אחדי' יש בה ו' מרובעי' כמו י"ו שרשו ד' כ"ה שרשו ה' ל"ו שרשו ו' מ"ט שרשו ז' ס"ד שרשו ח' פ"א שרשו ט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Every odd rank, such as the hundreds, which is third; the tens of thousands, which is fifth; the seventh, the ninth, and the like endlessly, is analogous to the rank of units, because there are also three squares in them. | וכל מעלה נפרד' כגו' המאו' שהי' השלישי' או הרבואו' שהם חמישית ושביעי' ותשיעי' וכן כלם דומי' דומי' למעל' האחדי' בשיש בם ג"כ ג' מרובעי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כגו' במאו' יש ק' ושרשו י' ת' ושרשו כ' ת'ת'ק' ושרשו ל' וכן כלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The squares in the odd ranks are always [analogous to] 1, 4, 9 and their roots are [analogous to] 1, 2, 3 in the preceding rank to its immediate right. | ולעול' א'ד'ט' מרבעי' במעלו' נפרדו' ושרשם א'ב'ג' מהמעלה שלפניה וימינה הקרובה לה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Every even rank is analogous to the tens. | וכן כל מעלה זוגית דומ' לעשרו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כגו' האלפי' יש בה ו' מרובעי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| As the squares in the tens are with addition of units, which is the closest rank to its right, so you always add to the even rank units from the preceding rank, which are added as units of the tens. | וכמו שבעשרו' המרובעי' עם חבור אחדי' מה שהיא המעל' הקרובה לימינ לפניה כן תחבר לעולם עם המעל' הזוגיי' אחדי' מהמעלה שלפניה במספר שתצטרך להוסיף אחדי' ע' העשרו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כגו' באלפי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
יש אלף ות"ר הדומ' לי"ו ושרשו ת'מ' והו' דומ' לד' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אלפים ות"ק הדומ' לכ"ה ושרשו נ' הדומ' לה' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ג' אלפי' ות"ר הדומ' לל"ו ושרשו ס' הדומ' לו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וד' אלפים תת"ק הדומ' למ"ט ושרשו ע' הדומ' לז' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וו' אלפי' ות' הדומ' לס"ד ושרשו פ' הדומ' לח' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וח' אלפי' ק' הדומ' לפ"א ושרשו צ' הדומ' לט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Likewise for every even rank. | וכן כל מעל' זוגיית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| This is the rule: every square of an odd rank is [analogous to] 1, 4, 9, whose roots are 1, 2, 3; and the squares of every even rank are [analogous to] 16, 25, 36, 49, 64, 81, whose roots are 4, 5, 6, 7, 8, 9, but the rank of the roots is never the closest to it. | וזה הכלל כל מרובע של מעלה נפרדת הו' א' ד' ט' ושרשם א' ב' ג' וכל מעל' זוגיי' שרש מרובעיה ו"א ה"ב ו"ג ט"ד ד"ו א"ח ושרשם ד' ה' ו' ז' ח' ט' ואבל אי' מעלת השרשי' לעול' הסמוכה לה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| This is how you know the positional rank of the root: always look for an odd rank to shift the root from the square back to it as the number of ranks it is away from the units. | וכה תדע מעלת מקו' השרש לעולם ראה במספרי' מעל' נפרדת להשיב השרש רחוק מהמרובע אחורני' מספר מעלו' כמספר מעלו' שירחק מהאחדי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והמשל מצאנו ד' במעל' תשיעי' כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה ידענו כי זה המספר מרובע כי הו' מעלה נפרד' ודומ' לאחדי' ובאחדי' ד' הו' מרובע ושרשו הו' ב' א"כ גם זה שרשו ב' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומקומה של הב' ראויה להיות ממוצעת בין מעלת האחדי' ומעלת המרובע והיינו בחמשית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Also for an even rank, since you always have to add the units of the preceding rank to the square and you have to shift the even rank back and consider it as tens in an odd rank, do as mentioned above and write the root in the middle. | וכן מספרי' הזוגו' מאחר שצריך לך לעול' לחבר עם המרובע אחדי' מהמעלה שלפניו וצריך להשיב מעלת הזוג אחורנית לחשבה בעשרו' ויהיו שם במעלה נפרדת עשה ג"כ כנ"ל ושים השרש באמצע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כאלו תאמ' מצאנו ו'ג' במעל' ח' והי' זוגיי' ודומה לל"ו שבעשרו' שהו' מרובע ושרשו ו' א"כ גם זה שרשו ו' וצרי' להשים הו' בין תחלת המספר המרובע ובין האחדי' באמצע דהיינו במעל' ד' שהו' רחוק מהאחדי' ג' מעלו' וכן הו' רחוק מהמעל' הה' אשר שם החל מספר המרובע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Here is the procedure for knowing the approximate or real root: | והילך מעשה ידיע' השרש הקרו' או האמתי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Write the number whose root is required in one row, according to its ranks. | כתו' המספר הדרוש שרשו בטור אחד כפי מעלותיו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
המשל בזה שמטהו ה' מעלו' כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה ידעת מהנ"ל שזה המספר נפרד ודומ' לאחדי' ר"ל הה' ושרש היותר קרו' בה הו' ב' שהו' שרש ד' הקרוב לה לפניו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומקו' הב' כנ"ל הו' במעל' ג' תחת הג' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ראה מעתה כמה מעלו' עברו מהאחדי' עד מקו' הב' שהי' שרשך ושם החל לכתו' גלגלי' כמספר המעלו' ההם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כאלו תאמ' מהאחדי' עד הב' יש ב' מעלו' לכן נכתו' תחת הקו ב' גלגלי' מתחילי' תחת הב' בעצמ' והולכי' לשמאל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אח"כ נכתו' העול' מכפל ב' על ב' שם והו' ד' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אח"כ גרע הד' מהה' של מספר הדרוש וישאר א' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן כתו' א' ממעל לה' והעבר קו סביב הה' כי אי' בה צורך עוד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומעתה נשאר לדעת השרש היותר קרוב מהב' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה יצטרך לקחת לכל מה מספר שתוסיף על השרש כפל אותו מספר על פעמים זה השרש שהו' הב' במשלינו במעל' המאות וגם כפל המספר ההו' על עצמו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומופת זה כי אנחנו הנחנו מספר מרובע של ד' רבואו' א"כ שרשו ב' מאו' כמ' שראית ושרשו הוא מספר הצלע האחת מהמרובע בעצמ' כי ב' מאו' אמ' ארך על ב' מאו' אמ' רחב ימצא בם ד' רבואו' אמו' של אמה על אמ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונניח שנוסיף על שרש זה המרובע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נמצא שתשים ל' רצועו' כל אחת של מאתים אמ' ארך וא' אמ' רחב דהיינו רצועה אחת של מאתים על ל' ותחברנה בצלע אחד מהמרובע כאלו תאמ' למזרחו ויהיה המרובע אז ארכו יתר על רחבו ארכו ר"ל ורחבו ר' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אח"כ הוספת עוד רצוע' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וא"כ כבר הוספת על זה השרש כפל ל' על ר' פעמי' פעם א' המזרח ופעם א' הדרום | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועדיין חסרה קרן זוית בזה המרובע והי' הקרן דרומי' מזרחי' אשר לא תתמלא רק אחר שומך שם מרובע ל' על ל' ואז ישוב מרובע שוה הצלעו' שרשו ר"ל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Illegible | [...] והיוצא מזה [...] שתכפול מה שתרצה להוסיף עליו על עצמו אח"כ על ב' פעמי [...] לעשו' שיהא בא צריך להוסיף עליו כפל ד' על עצמו שהו' י"ו [...] פעמי [...] גם כפל ד' ל"ד שהו' ב' פעמי' י"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Illegible | ונשוב לכונתינו ונאמ' אחרי שמצאת [...] וכתבתם [..] על מקומ' גם חסרונ' מרובעה של הב' מה"ה ונשאר א' שוב מעתה הוסיף על השרש מהמספר הנשאר והו' א' ב' ג' ד' א' ככל מה שתוכל וזה הדרך תלך בו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Illegible | קח השרש שמצאת ב' פעמים ר"ל כפול אותו עם ב' אם הו' ג' כתו' ו' ואם ח' ו'א' ובדמיוננו הו' ב' לכן כת'[...] במעלתו ד' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וחלק מעתה המספר העליון על הד' וראה כמ' פעמי' ימצא ד' בא' האחרונ' עם הד' שלפניה כשתשיבנה אחורני' ויהיו י"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הוי או' ג' פעמי' ימצא וצריך לראו' אם ימצא ג"כ למלאת הקרן זוי' והו' ט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כי אם לא היה אפשר לא הייתי לוקח רק ב' והנה בדמיוננו נוכל לקח[ת] ג' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונכתו' מעת' ג' קדם הב' לימינ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ראה כמ' מעלו' מהאחדי' עד הג' והנה הי' אחת | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
לכן כתו' תחת הקו במעלה ב' דהיינו תחת הג' ההי' גלגל אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אח"כ כפול ג' על עצמו ויצא ט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כתבהו במקומו תחת הקו לשמאל הגלגל היחיד שעשית תחת הג' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שוב כפול הג' על | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכתבם במקומ' אחרי הט' תחת הקו כי הג' הי' ממעל' העשרו' והד' מהמאו' וכפל עשרו' במאו' יעלו אלפי' לכן תחל לכתו' הב' תחת מעלת האלפי' והא' תחת הרבואו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אח"כ גרע זה מהמספר העליון כל מין ממינו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דהיינו הא' מן הא' ולא ישאר דבר והב' מהד' ישאר ב' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולפי שלא נוכל לקחת אז הט' מהג' לכן נשיב א' אחורני' ונכתו' א' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונקח הט' מהא' המושבת אחורני' על הג' שהם י"ג וישארו ד' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן נכתו' ד' ונכתו' זה למעלה מהטור העליון איש איש על מעלתו כמו שתראה בדמיונינו זה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והעבר קו על ג"ד ועל א' כי אי' בם צרך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה מצאת שרש הקרו' ר"ל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עו' תרצה להגדיל המרובע והשרש כי יש עוד מספר רב מן המספר הראשון הדרוש כי נשאר לך עדיין א' ב' ד' א' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ושוב מעתה לכפול גם הג' בב' ויהיה ו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כתבה תחת הג' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומעתה חלק המספר הנותר מהדרוש על כפל כל השרש והו' ו'ד' דהיינו ת"ס כי השרש הו' ר"ל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וראה כמה פעמי' ימצא ד' בי"ד והנהו ג' פעמי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כתו' ג' לפני הג'ב' של השרש ותחת הא' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכפול מעתה ג' על עצמו ויהיה ט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כתבה תחת הקו ותחת הג' האחדית [ויהיה למלא הקרן זוית] ולפי שהג' אינ' רחוקה דבר ממעלת האחדי' ע"כ אין צרי' לשים שום גלגל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד כפול הג' בשרש ב' פעמי' שהם ו'ד' ותמצא ח'ג'א' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כתבם על מקומ' דהיינו שתתחיל אצל הט' למטה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ויהיה המספר היוצא מכפל ג' על עצמו ועל השרש פעמים ט ח ג א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה המספר גרע מאשר נשאר לך מהמספר הדרוש והו' א ב ד א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה חסר א' מא' לא ישאר כלום | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד חסר ג' מד' ישאר א' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולפי שלא נוכל לקחת ח' מב' צריך להשיב זו הא' אחורנית ולא ישאר כלו' בזאת המעל' ג"כ | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חסר ח' מב'א' ר"ל מי"ב ישאר ד' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועו' צרי' להשי' אחורני' א' מהד' נשאר ג' וכן תכתו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עו' חסר ט' מא"א והו' י"א וישאר ב' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכתבה במקומ' והעבר קו על א"ב ד"א כי אי' בם צורך עוד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ויהיה השרש הקרוב למספר אבגדה בדרוש גגב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ויהיה המרובע כמספר כל המרובעי' שהוצאת מהשרשי' מגגב אחרי התחברם והו' טחבדה והנשאר מהמספר הו ב"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תשוב לחבר ב"ג על המרובע יצא לך המספר הדרוש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| I will give you various rules, the knowledge of which is necessary for the root extraction procedure. | אך אמנם אתן לך כללי' מתחלפי' יש צורך בידיעתם במעשה השרשים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The first rule: when you extract the first root from the last digit you have, if it is in an odd rank, or in the preceding to the last, if it is in an even rank, you should write that root in the middle rank, as I have shown you and take as much as you can. If you cannot take anything from from that rank, and you take from the [rank] that is second to it, write it as far from the place, from which you take the quotient, as the first root is far from the units. So, you should write a zero in the place that precedes the first root, and before this zero you should write the result of the division. | הכלל הא' כשלקחת השרש הראשון מהמספר האחרון שבידי אם הו' מעלה נפרדת או מהקודם לאחרון אם היא מעלה זוגיית ראוי לכתו' השרש ההו' במדרגה האמצעי' כהראיתיך ותקח כל מה שתוכל ואם לא תוכל לקחת דבר מהמקו' ההוא הראשון ולקחת מהשני לו גם כן תכתבהו רחוק מהמקו' שתקח ממנו ראש החלוק כאש[ר] השרש הראשון רחוק מהאחדי' ולכן ראוי לכתו' גלגל במקו' שלפני השרש הראשו' וק[ודם] לזה הגלגל תכתו' היוצא מהחלוק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The second rule: when you divide again what remains of the number you have by double the root, do not take the last number from which you took it first, even if you see that it is possible to take from it, because when you take the root from it first, you already take from it everything that should [be taken from it]. | הכלל הב' כשתשוב לחלק הנשאר מהמספר שבידך על כפל השרש לא תקח המספר האחרון שלקחת ממנו ראשונה ואף כי מהמספר [שאחריו] ואף כי תראה כי האפשר לקחת בו מפני שכשלוקח ממנו השרש ראשונה כבר לו[קח ממנו כל הראוי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Division of sexagesimal fractions |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| When you wish to divide a number that consists of degrees, minutes and seconds by another number, smaller or greater: | [77]כאשר תרצה לחלק חשבון מעלות ודקים ושניים על חשבון אחר יותר קטן או יותר גדול | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Take the number of the bottom row, which is the number by which you divide the number of the upper row, and convert all into the lowest type of fractions in it. | תקח חשבון הטור התחתון והוא החשבון אשר עליו מחלק חשבון הטור העליון ותשבר הכל אל מין השבר היותר קטן אשר בו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| I.e. if the lowest fraction in it is seconds, convert all into seconds; if thirds, convert all into thirds and so on. | ר"ל שאם השבר היותר קטן אשר בו הוא שניים תעשה מהכל שניים ואם שלשיים תעשה מה מהכל שלשיים וכן על זה הדרך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Then, take the number of the top row, which is the number divided by the number of the bottom row, and convert all into the [type of] fractions, which is as far from the type of fractions, into which you converted the bottom [number], as the type you want to receive in the division is far from the degrees. | ואחר כן קח חשבון הטור העליון והוא החשבון אשר יחולק על חשבון הטור התחתון וישבר אותו אל מן השברים שיהיה רחוק ממין השברים ששברת בו התחתון כמו שיהיה מרחק המין שתרצה שיצא לך בחלוקה מן המעלות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| I.e. if you converted the number of the bottom row into seconds and you want to receive thirds in division, convert the number of the top row into fifths that are three ranks away from the seconds, as the distance of the thirds from the rank of the degrees; the result of division will be thirds. | ר"ל שאם שברת חשבון הטור התחתון מן השניים ותרצה שיצא לך בחלוקה שלשיים הנה תשבר חשבון הטור העליון למין חמשיים שהוא רחוק מן השניים שלשה מדרגות כמו מרחק השלשיים מגדר המעלות וזה יצא בחלוקה שלשיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If you want to be more precise, so that the result of division will be fourths, convert the top row into sixths that are four ranks away from the seconds, as the distance of the fourths from the rank of the degrees; then you will get fourths from the division. | ואם תרצה לדקדק עוד שיצא בחלוקה רבעיים תשבר הטור העליון לששיים שהוא רחוק מהשניים ארבע מדרגות כמו מרחק הרבעיים מגדר המעלות ואז יצא לך בחלוקה רבעיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| And so on this way. | וכן על זה הדרך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If you covert the bottom row into thirds and you want to be more precise, so that you will get fourths from the division, convert the number of the top row into sevenths that are four ranks away from the fractions of the bottom row, as the distance of the fourths from the rank of the degrees; then you will get fourths from the division. | וכן אם שברת הטור התחתון לשלשיים ותרצה לדקדק עד שיצא לך בחלוקה רבעיים תשבר חשבון הטור העליון לשבעים שהוא רחוק משברי הטור התחתון ארבע מדרגות כמו מרחק הרביעיים מגדר המעלות ויצא לך בחלוק רביעיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If you want to be more precise, so that you will get fifths from the division, convert the number of the top row into eighths that are five ranks away from the fractions of the bottom row, as the distance of the fifths from the rank of the degrees; then you will get fifths from the division. | ואם תרצה לדקדק עד שיצא לך בחלוקה חמשיים תשבר חשבון הטור העליון לשמניים שהוא רחוק משברי הטור התחתון חמש מדרגות כמו מרחק החמשיים מגדר המעלות ויצא לידך בחלוקה חמשיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| And so on this way. | וכן על זה הדרך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| This is enough for you. | ודי לך בזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Extracting roots of sexagesimal fractions |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If you wish to find a root of any number you have consisting of degrees, minutes and seconds as many as they may be. | וכן אם תרצה למצוא שרש אי זה חשבון שיהיה בידך ממעלות הראשונים ושניים כפי מה שיהיה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Know up to which [type of] fractions you wish the root to be approximated, i.e. fourths, or fifths, or sixths, or which ever type of fraction you wish the approximate root to be composed of and denominate the root by it. | דע לך עד כמה שברים תרצה לדקדק שיצא בשרש ר"ל אם רבעיים או חמשיים או ששיים או אי זה מן שברים אשר תרצה לדקדק שיצא בשרש תקראהו מין השרש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Then, convert the whole number you have into the type of fractions, which is as far from the type [of the fractions] of the root as the [type of the fractions of the] root is far from the degrees. | ואחר זה תתיך כל החשבון אשר בידך אל מין השברים שיהיה רחוק ממין השרש כמו מרחק מן השרש מהמעלות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If the type [of the fractions] of the root is fourths, convert the number into eighths and the resulting root will be fourths. | שאם מין השרש רביעיים תתיך חשבון לשמוניים ומה שיצא השרש יהיה רביעיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If the type [of the fractions] of the root is fifths, convert the number into [tenths] and the resulting root will be fifths. | ואם מין השרש חמישיים תתיך החשבון לרביעיים ומה שיצא בשרש זה יהיה חמשיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| And so on this way. | וכן על זה הדרך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The rule of this method is that you multiply the type [of the fractions] of the root by its own name, one duplication: if its is seconds, convert them into fourths; if it is thirds, convert them into sixths; if it is fourths, convert them into eighths; if it is fifths, convert them into tenths. | והכלל בזה הדרך שתכפול מן השרש בשלו המראה לו כפלה פשוטה שאם יהיה שניים תתיך לרביעיים ואם יהיה שלשיים תתיך לששיים ואם יהיה רביעיים תתיך לשמוניים ואם הוא חמישיים תתיך לעשריים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Multiplication of sexagesimal fractions |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| When multiplying a number by a number, convert the top row into its lowest [type of] fraction. | אמנם בכפילת חשבון עם חשבון תתיך הטור העליון אל מין השבר היותר קטון אשר בו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Convert also the bottom row into its lowest type of fraction. | וכן הטור התחתון תתיך אל מין השבר היותר קטון אשר בו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The result of multiplication will be of the type of fraction denominated by the sum of the types of both rows. | והיוצא בכפילה יהיה מין השבר יקרא בשם המחובר המחובר משום הטורים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| I.e. if you multiply seconds by seconds, the result will be fourths. | ר"ל שאם תכפול שניים על שניים יהיה היוצא רביעיי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If seconds by thirds, the result will be fifths. | [78]ואם שניים על שלשיים יהיה היוצא חמישיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
A Rule for Checking the Squares |
כלל בבחינת המרובעים[79] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| When you have a number and you wish to check if it is a square, you can check it with the square that precedes it, or with the square that follows it: | כאשר יהיה בידך מספר ותרצה לבחון אותו אם הוא מרובע תוכל לבחון אותו עם המרובע הקודם לו לפניו ועם המרובע הקודם לו לאחריו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| First with the square that precedes it:
|
ראשונה עם המרובע הקודם לו לפניו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Consider the difference between the preceding square and the number you have. | תחשוב המרחק שבין המרובע שעבר ובין המספר אשר בידך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Divide the difference by double the root of the preceding square, the least possible by as little as you can. | חלק המרחק ההוא על כפל שרש המרובע שעבר פחת מן האפשר על המעט שתוכל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Subtract the quotient [and] the square of the quotient from the difference.
|
גרע מהמרחק ההוא היוצא מחלוקה וזה מרובע המספר היוצא מחלוקה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If the result of dividing the difference by double the preceding root plus the square of the quotient equals the difference you have, no more and no less, then the number is a square. Otherwise, you are wrong.
|
ואם היוצא לך מחלק המרחק על כפל השרש שעבר עם מרובע המספר היוצא מחלוקה שוה אל המרחק אשר בידך לא פחות ולא יתר הנה המספר מרובע ואם לא הנה טעית[80] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| You can check it also with the square that follows it: | תוכל לבחון גם כן עם המרובע הקודם לו לאחריו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Consider the difference between the number and the following square. | תחשוב המרחק שבין מספר ובין המרובע העתיד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Divide the difference by double the following square, and give it as much possible by as little as you can. | חלק המרחק ההוא על כפל שרש המרובע העתיד ונכון לו יותר על האפשר על המעט שנוכל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Subtract the difference and the square of the quotient from the quotient. | גרע המרחק ומרובע המספר היוצא מחלוקה מהעולה בחלוק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If the result of division plus the square of the quotient equals the difference, then the number is a square. Otherwise, you are wrong. | ואם העולה מהחלוקה עם מרובע המספר היוצא מחלוקה שוה למרחק הנה המספר מרובע ואם לא הנה טעות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Over and done | תם ונשלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Praise be to the Lord of the world. | תהלה לאל עולם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| When the analogous square is less than the required number, we must take the greatest possible quotient so that the quotient is [equal to] the difference minus the square of the quotient.
|
כאשר יהיה המרובע הנמשל פחות מהמספר המבוקש צריך שנקח החלוק מספר היותר גדול שנוכל שיעלה בחלוקה המרחק כאשר יחוסר ממנו מרובע מה שיעלה בחלוק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| When you add the product to the analogous square with the square of the quotient, it is the square closest to the required number.
|
וכאשר תוסיף על המרובע הנמשל כמו הכפל עם מרובע מה שיעלה בחלוק הוא המרובע היותר קרוב על המספר המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| When you add the quotient to the root of the analogous square, it is its root.
|
וכאשר תוסיף על שורש המרובע הנמשל מספר מה שיעלה בחלק הוא שרשו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| When the required number is less than the analogous square, we must take the smallest possible quotient so that the quotient is [equal to] the sum of the difference and the square of the quotient.
|
וכאשר היה המספר המבוקש פחות מהמרובע הנמשל צריך שנקח החלוק מספר היותר קטן שנוכל שיעלה כמו המרחק מחובר עם מרובע מה שיעלה בחלוק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| When you subtract the product from the analogous square [and add] the square of the quotient, it is the square closest to the required number.
|
וכאשר תגרע מהמרובע הנמשל כמו הכפל כאשר יחובר ממנו מרובע מה שיעלה בחלוק הוא המרובע היותר קרוב אל המספר המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| When you subtract the quotient from the root of the analogous square, it is its root.
|
וכאשר תגרע משרש הנמשל מספר מה שיעלה בחלוק הוא שרשו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The difference it the difference between the analogous square and the required number. | המרחק הוא המרחק שבין המרובע הנמשל ובין המספר המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The product is the product of the quotient by double the root of the analogous square.
|
הנכפל הוא מה שיעלה מכפלת מספר מה שיעלה בחלוק עם כפל שורש המרובע הנמשל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Because, when there are two different squares, the ratio of the product of one root by the other to the first square is the same as the ratio of the second square to the product.
|
כי יהיו שני מרובעים מתחלפים יחס המחובר מכפלת אחד מן השרשים על האחר אל המרובע הראשון כיחס המרובע השני אל המחובר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
MS London 7r-v |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| He said: the reasons of the division are two: | אמר טעם החלוק הם שניים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
א שנכפול בדרך הכפל מה שיצא בחלוק על הטור השפל שמחלקים עליו ונחבר עם העולה מה שנשאר לחלק אם נשאר דבר ואם המחובר שוה למספר הטור המחולק עשינו נכונה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הב' שנכפול מאזני מה שעלה בחלוק עם מאזני הטור השפל ונקח ממנו העולה ואם נשאר דבר לחלק נחבריהו עמו ואם הוא שוה למאזני הטור העליון חשבונינו אמת | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The reason for the first scales is that the dividend number has as many times the divisor as the result of division, which is a number that counts the divisor in the greater number, which is the dividend. Therefore, [the product of] this by that is the dividend. | והטעם למאזנים הראשונים כי בחשבון המחולק מדמיוני החשבון שמחלקים עליו כשיעור אחד מה שיעלה בחלוק הוא מספר מה שימנה המספר שמחלקים עליו למספר גדול המחולק לזה ככה זה על זה ויצא המחולק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Chapter on Summation |
שער המחברת | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| To know how much is the sum of numbers from a known number to the last number. | לדעת כמה המחובר מהמספרי' ממספר ידוע עד סוף מספר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כאלו תאמר רצינו לדעת המחוברים מאחד עד עשרה כמו א' עם ב' הם ג' ג' עם ד' הם ז' ותרצה לדעת בקצור | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם הם זוג כמו עשרה ונוסיף עליו א' ותקח חצי העשר שהם חמשה וכפול החמשה על הי"א והוא המספר האחרון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם נפרד כמו מאחד עד שבע תוסיף על ז' אחד ויהיו ח' ותקח חצי המספר והם ד' וכפול הד' על הז' והוא המכוון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Do this with any number, small of large, even or odd. | וכן תעשה בכל מספר קטן או גדול זוג א[ו] נפרד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם תרצה לדעת ערך נעלם כמו שתאמר ערך ד' אל ו' כערך ח' אל הנעלם ותרצה לדעת | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
האמצעיים ו' על ח' יהיו מ"ח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותחלק המספר הראשון שהוא ד' על המ"ח ויעלה לך חלק מ"ח והוא הנעלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Abraham said: I have found another way: add to the square of the last number its root, then take half of the result; that is the sum. This way helps to understand the inverse question.
|
אמר אברהם מצאתי דרך אחר שהמוסיף על מרובע סוף החשבון שרשו ולוקח חצי בעולה שם ימצא המחובר והדרך הזה יורה להבין הפך השאלה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון שאל שואל חברתי מספרים עלו תס"ה כמה יהיה סוף החשבון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| This is the rule: take the sum, always double the sum, then take the root of the square, i.e. the preceding square, and check it: if between the square and the double remains the root no more and no less, know that the root is the required.
|
כלל זה תקח בידך היוצא [...] כפול לעולם החשבון המחובר והשורש תקח מן המרובע כלו' פי' הנכפל שעבר ובחן אותו כ"א נשאר בין המרובע ובין הנכפל כמו שורש בלי תוספת ומגרעת תדע כי החשבון והשורש הוא המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה כפלנו תס"ה על(ה) תתק"ל ידענו כי מרובע שעבר הוא ט מאות ושרשו ל' שהם המספרי' המרובעי' ואין בין המרובע והנכפל כ"א ל' ועל כן תבין כי נכון מה שחשב והמבוקש ל' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Word Problems |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
"How Many" Problem |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שאלה אדם עבר על | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נקח להם אחד נוסיף להם עוד אחד הנה נוסיף להם מחציתו הנה שנים וחצי נוסיף רביעיתו יהיה ג רביעי' ובעבור שהם רביעיות נוספות על השלמי' נשים גם השלמי' לרביעיות ויהיו ה וג' רביעיות הנה י"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ובעבו' כי אמר שיהיו יהיה מספרם עם התוספת ט"ט לבד אותו שדבר עמהם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נחלק הט"ט שברים לרביעי' ויהיו שצ"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נחלקם על י"א יהיו ל"ו וככה מספרם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
"If You Give Me" Problem |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שאלה ארבעה קונים חפץ אחד אחד אמר לחבירו כל אחד יתן החצי אשר בכיסו ואני אתן כל מה שיש בכיסי ואקנה החפץ | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ערך החפץ מ"ו דינרי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
לראשון ט"ו פשוטי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולשיני כ"ג דינרי' וט' פשיטי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
לשלישי ל"א דינרי' ג פשיטי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
לרביעי ל"ה דינרי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ע"א להגיע עד חצי שליש ורביע החפץ י"ז פשיטי' לאחד ה' לשנים י"א לשלישי י"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
"Find the Amount" Problem |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שאלה אדם מכר י"ג מדות בכ"ג פשי' כמה מדות יתן בז' פש' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ערך ז' אל כ"ג כן ערך הנעלם אל י"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נכפול הקצוות שהם נודעים יהיו צ"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נחלקם על כ"ג יעלו ג' מדות וכ"ב חלקי' מכ"ג במדה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| "Find the Price" Problem | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
|
ועוד נהפך הענין שנרצה לדעת בכמה יתן לו ז' מדות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה נעשה הדמיון הזה נכפול הקצוות יהיו קס"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נחלקם על י"א והיו י"ב פשיטי' וה' חלקי' מי"ג בפשיט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם שאל בכמה יתן ז' מדות יהיה ערך הנעלם בכ"ג אל כ"ג כערך ז' אל י"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Pursuit Problem |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שאלה אדם הולך בכל יום כ"ט מילין אחר י' ימים נסע השני ההולך בכל יום ל"ז מילין | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפול המילין שהולך בי' ימים יהיו ר"צ | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נחלקם על היתרון שיש בין שני המהלכים שהוא ח' והנה ל"ו ימים ורבע יום | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה כערך י' ימים אל הנעלם כן ערך היתרון שהוא ח' אל כ"ט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Encounter Problem |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שאלה בכמה ימים יכלה מהלך ק' על ידי שני מהלכים של י"ט ושל י"ז זה לקראת זה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וקל לדעת כי הוא כערך מחובר שניהם אל קו וחלקם עליו יהיה ב' וכ"ח מל"ו כמו כן בב' ימים וכ"ח מל"ו ביום יכל ישהו לכלות וכ"ח מל"ו הם ז' תשיעיות היום | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תרצה לדעת כמה שעות הם ידוע הוא כי שעות היום י"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה ז' אל ט' כן ערך הנעלם אל י"ט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Payment Problem |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שאלה אדם עם ג' שיתן לראשון ה' זהובים ויעבוד לו כ' ימי' ולא רצה לגמור כל העבודה ואמר לשיני שיתן לו ד' ולא רצה לגמור | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה זה דומה לשאלת המחליף אך נשים הזהובים המחולקי' לפועלי' במטבעות והעבודה הנשכרת היא הזהוב והמורה הוא ס' ומחובר החלקי' מ"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה כערך מ"ז אל ס' כן ערך הזהוב אל הנעלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה החלק השוה זהו' אחד וי"ג ממ"ז בזהוב אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נבקש ערך כל אחד ב' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה בעל הג' הם ו' ימי' וב' שלישיות היום | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ובעל הד' בזהו' אחד ה' ימים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ובעל הה' בזהוב אחד ד' ימי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה של ג' עובד בשביל ח חלק העולה לו במ"ז כערך י"ג אל מ"ז כן ערך הנעלם אל כ' שהוא מספר שעות עבודתו בזהו' אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ושל ד' כערך י"ג אל מ"ז כן ערך הנעלם אל מ"ח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תחבר כל העולה בזה יעלה המחובר כ ימים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Boiling Problems |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שאלה אדם אמר לבשל עשרה מדות מתירוש עד השאיר שליש לאחר שנתמעטו ב והיו ח נשפך ב והיו ו ושאל השואל כמה יהיה ראוי לישאר מן העומד לכ' לפנינו אם יבא לבשלו עד שיהיה כמשפט הראשון לפי מה שהתחיל כי ידוע שנתמעטו מג' ושליש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה כערך ו' אל ח' כן ערך הנעלם אל ג' ושליש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Whole from Parts Problems - lance |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שאלה רומח מחציתו שלישיתו במים רביעיתו בעפר וגלוי ז אמות כמה גבהות כלו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה המורה י"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נכסה ממנו חלקיו אלה יהיה הגלוי ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה כערך ה' אל י"ב כן ערך ז' אל הנעלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Multiple Quantities Problems |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שאלה אדם צוה לתת כ' פשוט לכ' בני אדם אנשים ונשים ובנים לאיש נתן ב' פשיט ולאישה מחצה ולבנים [...] כמה לאיש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
לח' אנשי' יו פשיטי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
לח' בני' ב' פשיטי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
לד' נשי' ב' פשי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שאלה אדם אמר לעבדו קח ל' פשיטי' וקנה לי ל' עופות אווז ב' פשיטי' שליו מחצה פרגיות פשיט כמה יהיו מכל מין | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ו' פרגיות ח' אווזות י"ו שלוים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Whole from Parts Problems - fish |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שאלה דג יש לו טו אצבעות בגודל גופו לבד ראשו וזנבו וראשו מחזיק שלישית כל הדג והזנב מחזיק רביעיתו כמה מחזיק כלו עם הראש והזנב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ל"ו אצבעות שלישיתו י"ב והרביעית ט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפל החשבון שלישי' ורביעי' ותמצא כך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שאלה גנב הולך סכום אחד והרודף יום ראשון א ויום שיני ב וכן ויוסיף כל הימי' ב' כאשר יגדלו הסכום כפל הגנב ובתוך כך תפל אחד ובתוך כך סכום ימים ישיג הגנב בכל סכום שילך כמה בכל יום | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שאלה שמעון הרויח שביעית ממנו היום ולמחר הרויח מן הכל חמישית ויום שלישי מן הכל שלישית והתחבר י' דינרי' ונשאל כמה הממון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
המורה ק"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שאלה לשקול ביא אבני' ס' [..] גם בי"ח טבעות לשכירות לד שנים וב חדשי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
יצא בכפל למשקל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן תעשה בטבעות א ב ד ח י"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכפול עד שיעלה החשבון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ובאבנים יצא בשלישות א ג ט כז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Glosses on Abraham Ibn Ezra’s Book of the number (P1026) |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
The author of the Sefer Yezira |
ובעל ספר יצירה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Explanation: The intention of the author of Sefer Yezira [= the Book of Creation] is to inform how man was created by one act from the creatures. | פי' בעל כוונת ספר יצירה להודיע איך נברא האדם בתחבולה אחת מין הנבראים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| He said that three matters were needed for this: | ואמ' שיצטרכו בו ג' עניני' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
SefarThe writing: through the faculties of the letters that are indicating him, the creation is done by the letters. |
הספר הוא הכתיבה כי בכחות האותיות יורו בו באותיות יעשה היצירה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
SeferIt is the number, because composition and merging are only done by that one number is the duplication of the other. |
וספר הוא המנין כי ההרכבה והמזגה לא תעשה רק במספר שבאחד מהם יהיה בו מיסוד האחד כפל האחר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
SipurBecause the scholars of this science assumed that they were leading down a spiritual force that gave power to that composition, so that the action of this power is not through anything, but not through anything is possible only through God. |
וספור כי חכמי חכמה זו הניחו שהם יורידו כח אשר נתן כח בהרכבה ההיא עד שיעשה פעל הכח בלתי מדבר אבל לא מדבר כי זה לא יתכן אלא לאל ית' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Since |
בעבור | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The word "since" is applied until his saying below, "Therefore, the scales of every number". | מלת בעבור נמשכת עד אמרו למטה על כן כל מאזני כל מספר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Sefar is the composite. | ספר הוא המרוכב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Sefer is the number, as "after the numbering wherewith [David his father] had numbered them [Chronicles 2, 2, 16]. | וספר הוא המספר כמו אחר הספר אשר ספרם[note 1] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Sipur is the expression of units. | וספור הוא הדבור באחדים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Higher decade |
בכלל הגבוה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Meaning: the closest to it [above] it. | פי' הקרוב לו לפניו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
The remainder is the required |
והנשאר הוא המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Explanation: a third multiplied by a third is a ninth.
|
פי' שהשלישית הנכפל על השלישית יצא תשיעית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Therefore, when we multiply a third of a number by its third, we receive a ninth of the required square.
|
אם כן בכפלנו שליש המספר על שלישו יצא לנו תשיעית מרובע הדרוש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| When we take the same in the higher rank, we receive ten times the ninth of the square. | וכאשר לקחנו כמוהו בכלל הגבוה ממנו יצא לנו עשרה דמיוני תשיעית המרובע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Therefore, when we subtract from it the square of the third, which is a ninth of the square, the remainder equals the required square. | אם כן כשחסרנו מזה מרובע השליש שהוא תשיעית המרובע ישאר שוה למרובע הדרוש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
The sum is the required |
והמחובר הוא הדרוש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
פי' אעפ"י שלא זכר המחבר דרך זו רק בהוצאת המרובעים יתכן זה בכפל כל שני מספרים בעלי שליש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כמו י"ב וי"ח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אנו נכפול שליש האחד והוא ד' בשליש השני והוא ו' ויעלה כ"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נגביההו מעלה אחת ויהיה ר"מ | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הסיר [81]כפל השני שלישיות שהוא כ"ד ישארו רי"ו והוא כפל י"ח על י"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם שהם נעדרי השליש ומוסיפין אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כמו י"ג י"ט נעש[ה] כן | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נוציא סך החשבון לי"ב וי"ח ויעלה רי"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולפי שכל אחד מהמספרים מוסיף אחד על השליש נוסיף י"ב וי"ח ואחד ועלה רמ"ז וזהו כפל י"ג בי"ט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם היו שני המספרים חסרין אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כי"א וי"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נעשה החשבון בי"ב וי"ח ויעלה רי"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ובעד חסרון האחד נגרע י"א וי"ז ואחד מן רי"ו וישאר קפ"ז והוא כפל י"א בי"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם האחד מוסיף אחד והשני חוסר אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כי"א וי"ט או י"ג וי"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נעשה החשבון בי"ב וי"ח ויעלה רי"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה אם היו י"א וי"ט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
התוספת הוא י"א והחסרון הוא י"ח אם כן החסרון רב על התוספת ז' חסרם מן רי"ו וישאר ר"ט והוא הדרוש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם היו י"ג וי"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה התוספת הוא י"ז והחסרון י"ג אם כן התוספת רב ה' נוסיפם על רי"ו והיה רכ"א והוא הדרוש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם הן כמו י"ח וי"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נוסיף על רי"ו י"ח והיה רל"ב והוא הדרוש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם הן י"ח וי"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נגרע י"ח מן רי"ו וישאר קצ"ח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Example: we wish to multiply 29 by 31 |
דמיון רצינו לכפול כ"ט על ל"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
פי' דמיון אחר ברצותנו לכפול כמשל ח' על י"ב שמרחקם מי' שהוא כלל מספר שוה והוא ב' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נכפול י' על עצמו והיה ק' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נחסר ממנו ד' שהוא מרובע ב' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ובאור זה שכפל ח' על י"ב הוא כפל ח' על י' ועל ב' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכפל י' על י' הוא כולל כפל י' על ח' ועל ב' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכפל י' על ב' הוא כולל כפל ח' על ב' וב' על ב' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נמצא יתרון י' על י' מן ח' על י"ב הוא ב' על ב' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| From this you understand all the other examples, and this is according to proposition 6 of Euclid's Book II. | ומזה תבין לכל המשלים האחרים וזה מתמונת ו' ממאמר ב' לאיקלידיס | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The rule is the same for any two different numbers, even if there are no decades: we add to the smaller number half the difference between the two numbers, square [the sum], subtract from the square the square of half the difference and the remainder is equal to the product of one of the two numbers by the other.
|
וכן ההנהגה בכל שני מספרים מתחלפים אך כי לא יהיו כללים שנוסיף על המספר היותר קטן חצי יתרון שבין שני המספרים ונרבעהו ונחסר מהמרובע מרובע חצי היתרון והנשאר ישוה להכאת אחד משני המספרים [82]באחר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| But this scholar wanted to make it easier, not harder, and for this reason he explained it only for the numbers whose distance from a decade is the same. | אך זה החכם בא להקל ולא להחמיר ולזה לא באר במספרים שמרחקן מחשבון כלל מספר שוה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Know that if there are two digits to multiply |
ודע כי אם יהיו שני מספרים לכפול | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Explanation: if you wish to multiply 2 by 40, it is just a multiplication of one rank by another, but if we have one digit by two digits, or two by two, the ranks must be multiplied by each other according to the numerical value of the digits. | פי' כי אם תרצה להכות ב' על מ' אין כאן הכאה רק ממדרגה האחת על האחרת אך אם יהיה לנו מספר על שני מספרים או שנים על שנים אז צריך לכפול המעלות זו על זו כפי מנין המספרים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
You have to |
אתה צריך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Explanation: he said it in actu, although sometimes it can be done with less, because he said above: if they are two by two, it should be done 4 times, and then he explained that it can be done with less, so here too it can be done with less. Understand. | פי' אמ' זה בשלוח אעפי שלפעמים יתכן בפחות מזה כי כן אמר למעלה ואם הם שנים על שנים צריך לעשות זה ד' פעמים ואחר כן באר שאפשר בפחות וגם בזה אפשר בפחות ובין | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
As if they are units |
כאלו הם אחדים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Explanation: the reason for this is that every decade exceeds by one over the ninths, therefore we have to consider each decade as one. | פי' וסבת זה כי כל כלל יוסיף אחד על תשיעיות וכן שנים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The reason for this is that the foundation of number are 9 numbers and here their units are inclusive. This is his statement at the beginning of the book: "therefore the scales of the number are 9". | וסבת זה היות ט' מספרים יסוד החשבון והכא אחדיהם הוא כולל והוא אמרו בראש הספר על כן היו מאזני המספר ט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Does not assume any change |
לא יקבל שנוי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Explanation: because change comes from composition, and the one is simple from being one. | פי' כי השנוי יבא מצד ההרכבה והאחד פשוט כי מהיותו אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Does not assume any increase |
לא יקבל רבוי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Because 1 [multiplied] by 1 is 1. | כי א' על א' הוא א' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Does not assume any division |
ולא חלוק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Because, if you divide it, there are two. | כי אם תחלקהו יהיו שנים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
It is the cause of any change |
והוא סבת כל שנוי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Because the change results from the combination of two matters and the same for the number. | כי בהרכבת שני ענינים יבא השנוי וכן במספר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
According to its rank |
כפי מעלתו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Explanation: units corresponding to units and tens corresponding to tens. | פי' אחדים כנגד אחדים ועשרות כנגד עשרות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Less than the number |
ופחות מהמספר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Explanation: the dividend number should be greater than the divisor number, because it is like the measure and the measured. | פי' שהמספר המחולק ראוי להיות יותר גדול מהמספר המחלק כי זה כמו המדה עם הנמדד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| More precisely, this is not mandatory, as will be explained regarding the division of fractions, when we divide five-ninths by a whole number. | וזה ביותר נכון לא מחויב כמו שיתבאר בחלוק השברים שנחלק חמש תשיעיות על שלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Did not reach the rank of the units |
ולא הגיע למעלת האחדים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Explanation: the result of division has not yet reached the rank of the units, because, if it had, it would have already been completely gone and would no longer be subject to division, since the dividend has already been divided into a number smaller than the divisor. | פי' שהיוצא בחלוק לא הגיע למעלת האחדים שאם היה כן כבר יצא לחוץ ולא יקבל עוד החלוקה שכבר נתך המחולק אל מספר יותר קטן מהמחולק עליו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| By saying: not subject to division, i.e. for integers, but for fractions, it is subject to division. Understand. | ובאמרנו לא יקבל החלוקה ר"ל בשלמים אך בשברים יקבל החלוק ובין | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Not gone completely |
[83]כי לא יצא לחוץ | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Explanation: decomposing the dividend until it reaches a number smaller than the divisor, is called completely gone [lit. exiting]. | פי' התכת המחולק עד שיגיע למספר יותר קטן מהמחולק עליו יקרא יציאה לחוץ | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If it is divided [..], it reaches outside the rank of units, therefore it becomes fractions, ten of which is one whole, and they are called primes. Every prime is divided into 10 seconds. He did not aim only to the division of integers by integers. | שאם נחלק [..]יצא לחוץ ממעלת האחדים ויהיו שברים אשר העשרה מהם הוא אחד שלם יקראו ראשוניים וכן כל ראשון יחלק לי' שניים והוא לא כיון רק בחלוק שלמים על שלמים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
We always subtract backwards |
ולעולם נחזור אחורנית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| It is necessary to investigate what brought him to this, because it is more correct and easier to subtract directly. | צ"ע מה הביאו לזה כי יותר נכון ונקל לחסר ביושר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
One is like a point |
האחד כנקדה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Explanation: because the point is like the foundation of the circle because the one who draws a circle, before drawing it, draws a center, on which he establishes the circumference of the circle. | פי' כי הנקדה כיסוד לעגול כי המקוה לעגול טרם הקוותו יניח מוצק עליה יכונן רחב העגול | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The one is the foundation of the number, and therefore it cannot be divided like the point, since it is one. | וכן האחד הוא יסוד המספר ולכן לא יתכן לחלק כמו הנקדה מצד שהוא אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| But since every sum has the name of one, being composed of many individuals, like the body, which is composed of four phlegms and other organs, which have homogeneous parts, and the hand and the arm, and the rest of the organs, hence it is undoubtedly possible for it to be decomposed into parts, except for the intellect, in which the speech is created, for this reason it was decided that the simple division is done in the intellect, because without the intellect it is impossible in any way. | ואולם מצד ההוה כל כלל בשם אחד עם היותו מורכב מאישים רבים כמו הגוף המורכב מד' ליחות ומשאר אברים מתדמי החלקים ומהיד והזרוע ושאר אברים הכליים והנה הוא בלי ספק יתכן בו התפרקו לחלקו חוץ לשכל יתחדש בו הדבור בעבור זה החליטו החלוק הפשוט להעשותו בו בשכל כי חוץ לשכל אי אפשר בשום פנים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Another explanation: all this is an explanation for dividing the one into fractions, and just as the point is an indivisible thing and all lines are drawn from it, so is the one, and for this reason it cannot be divided. | עוד פי' כל זה הצעה לחלוקת האחד לשברים וכמו שהנקדה היא דבר בלתי מתחלק וכל הקוים יוצאים ממנה כן האחד ולזה לא היה ראוי לחלק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Consists of surfaces |
מורכב משטחים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Explanation: he did not mean that the body is a chemical composition of surfaces and not of lines and points, as explained in the physics books, but in his statement he meant the surfaces of the encompassing objects and many in the human body, such as the skull that is encompassed by one surface, and so are many organs, and the body is called one. | פי' אין כונתו שהגוף הרכבה מזגיית משטחים ולא מקוים ונקדות כמבואר בספרי הטבע אך כונתו באמרו שטחים התכליות המקיפות ורבים בגוף האדם כמו הגלגלת יקיפהו שטח אחד וכן אברים רבים והגוף יקרא אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
It is similar to an odd number |
והוא דומה לנפרד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Explanation: when you sum up the odds in sequence the squares of the number of the odds are generated. | פי' שכשתחבר הנפרדים על הסדר יולידו מרובעי מספר הנפרדים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Because 1 is the square of 1.
|
כי א' הוא מרובע א' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 1 and [3] is the square of 2.
|
וא' עם הוא מרובע ב' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 1, 3 and 5 is the square of 3.
|
וא' עם ג' וה' הוא מרובע ג' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| This is one of the properties of the number. | וזה אחד מסגלת המספר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
It is a half of an eighth |
הוא חצי שמינית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If you divide the product by the square of the denominator, the result of division is an integer. | אם תחלק הנכפל על מרובע המורה יהיה היוצא מהחלוקה שלמים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If it cannot be divided, i.e. the square of the denominator is greater than the product, or it can be divided, but there is a remainder that cannot be divided, they are fractions named by the name of the square. | ואם לא יתחלק ר"ל [84]שיהיה מרובע המורה יותר גדול מהנכפל או יתחלק וישאר שלא יתחלק יהיו שברים נקראין בשם המרובע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If you divide the product by the denominator, the result are fractions named by the name of the denominator and what cannot be divided are fractions of fractions named by the name of the denominator. Understand. | ואם תחלק הנכפל על המורה יהיה היוצא שברים נקראין בשם המורה ומה שלא יתחלק יהיו שברי שברים נקראין בשם המורה ובין | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Let this rule be handed down to you: after you know the denominator, its square, and the product, divide the square by the product, or divide the product by the denominator. | ויהיה כלל זה מסור בידך אחר שתדע המורה ומרובעו והנכפל תחלק המרובע על הנכפל או תחלק הנכפל על המורה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
But I shall teach you a short method |
רק אלמד דרך קצרה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Explanation: because according to what we said above, one denominator should be produced for all of them by multiplying one by the other, because the reason for the denominator issue is to find a number that will have all of these parts. | פי' כי לפי מה שאמרנו למעלה היה צריך לעשות מורה אחד לכלן בכפל האחד על האחר כי הסבה מענין המורה הוא למצא מספר שיהיו בו כל חלקים אלו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד באמת לא היה צריך לקחת ששיים כי די לו בשלישיות וזה נכפול על ה' יהיו ט"ו עוד נכפול זה על ז' יהיו ק"ה עו' נכפול זה בח' יהיו תק"מ והוא המורה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נבקש המספר ראשון שהוא ב' שלישית רביעית החמשית יהיו כ"ח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כי החמשית הוא קס"ח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ורביעיתו מ"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ושתי שלישיותיו כ"ח וזהו החשבון הראשון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וידענו ששמיניתו ק"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ושביעיתו ט"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והשש שביעיות הם צ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נכפול כ"ח על צ' יעלה אלפים ת"ק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקנום על תת"מ שהוא המורה ויעלה ג' שהן חלקים מהתת"מ שלמים והן חמשית שביעית השמינית שהשמינית ק"ה ושביעיתו ט"ו וחמישיתו ג' והוא הדרוש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| It is necessary to examine the example that the scholar chose, because it is more appropriate to take the example with the smallest possible number. | וצ"ע בדמיון שלקח החכם כי יותר ראוי לקחת הדמיון בקטן המספר שאפשר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| It is also necessary to examine why it was necessary to explain that since we have sixths, there is no need for a third as if the sixth had to be divided. | גם צ"ע למה הוצרך לבאר שאחר שיש לנו ששיות אין צריך לשלישית כאלו היה צריך לחלק ששית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
The difference between the fifth and the ninth is four |
החמשית והתשיעית ארבע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| It is necessary to examine how he used the first method, because it does not apply to all numbers: | צ"ע איך לקח דרך זה הראשון כי לא ימשך בכל המספרים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה שנאמר לקחנו שלישית הממון וחמשיתו ושביעיתו כמה הוא מערך הממון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולפי דרכו יהיה כן | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נחשב כאלו הן ג' שביעיות שהוא השבר היותר פחות אחר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נכפול מה שבין השביעית והשלישית [85]שהוא ד' על מה שבין החמישית והשלישית שהוא ב' ויעלה הכפל ח' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונעשה מהחמשה שביעית אחת ויהיו ד' שביעיות וישאר ג' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונחברם עם ב' שהוא היתרון שבין שלישית לחמישית והיה ה' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולפי זה יהיה הערך ה' שביעיות ויעלה ע"ה לפי שהמורה ק"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולפי הדרך שני שהוא האמתי יהיו ע"ה חלקים מן ה' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| It is also necessary to examine how he said in his example that the three are 3-fifths of a seventh of a ninth, when he initially said: we convert the 6 into a ninth. | עו' צ"ע איך אמ' בדמיונו שהשלשה היו ג' חמשיות שביעית התשיעית והוא אמר תחלה נעשה מן הו' תשיעית אחת | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| There is another correct way to do this, only the increment between the [denominators of the] fractions is the same, such as 4, 5, 6, or 5, 7, 9, and it is to take the type of the middle fraction, instead of the three types of the three fractions, then the difference between the extremes is multiplied by the increment, and the sum is the required. | ויש בזה דרך אחרת נכונה רק שיהיה הדלוג בשברים שוה כמו ד'ה'ו' ה'ז'ט' והוא לקחת מקום הג' מינין ג' שברים ממין האמצעי ואחר יוכפל מרחק הקצוות כמרחק הדלוגים והמחובר הוא הדרוש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Example: The ratio of 4 to [6] |
דמיון ערך ד' וה' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
פי' וזה יתבאר כי מרובע הקו כלו שהוא י"ו שוה לשני מרובעי י"ב וד' בתמונת ד' מב' לאקלידיס | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ושטח ו' בח' שוה לשטח שיקיפו בו י"ב וד' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
לפי שיחס ד' אל ו' כיחס ח' אל י"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם כן מרובע י"ו שוה לשני מרובעי י"ב וד' ולכפל השטח שיקיפו בו ו' וח' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אבל שני מרובעי ו' ח' שוין לכפל השטח שיקיפו בו ו' ח' עם מרובע מה שבין ו' ח' כמבואר בה' מב' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם כן מרובע י"ו עם מרובע היתרון שבין ו' לח' שוה לד' מרובעי ד' ו' ח' י"ב ובזה יתבאר מהשני והשלישי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Its proportions are composed |
כי ערכיה מורכבין | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Explanation: the harmonic proportions consist of the geometric and the arithmetic proportions, as they are determined by the division of the excess of the one by the other and the ratio of one number by the other number.
|
פי' ערכי הנגונים הן מורכבים ערכי המדות וערכי המספר כי יעשה בחלק היתרון האחד על האחר וכיחס המספר האחד אל המספר האחר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Because the ratio is always |
כי לעולם יהיה הערך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Because the ratio of what is between the first and the mean to what is between the mean and the last is always the same as the ratio of the first number to the last number. | כי לעולם יהיה היחס מה שבין הראשון והאמצעי אל מה שבין האמצעי והאחרון כיחס המספר הראשון אל האחרון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Question: Reuven hired Shimon |
שאלה ראובן שכר שמעון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הכלל בכל דרכים אלו שנכפול י"א על ט' ויהיו צ"ט וחלקם על י"ז ועלה ה' פשו' וי"ד חלקים מי"ז [86]בפשו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| This rule is given to you in proportions: the number that corresponds to the unknown, which is in the place of the zero, is the denominator. If zero is one of the extremes, the other extreme is the denominator; and if zero is one of the means, the other mean is the denominator. | כלל זה יהיה בידך בערכין שאותו המספר שיהיה גיל הנעלם שהוא מקום הגלגל מן המורה שאם היה הגלגל אחד מהקצוות הקצה האחר הוא המורה ואם הגלגל הוא אחד מהאמצעיים האמצעי האחר הוא המורה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
And you do not know the third: multiply the first by the second |
ולא תדע השלישי כפול הראשון על השני | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| This is clear from 19.V: when the sum to the sum is as the ratio of the whole to the whole, then the ratio of the remainder to the remainder is as the ratio of the whole to the whole. | זה יתבאר מי"ט מה' באמת כאשר היה המחובר אל המחובר כיחס הכל אל הכל הנה יחס הנשאר אל הנשאר כיחס הכל אל הכל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולכן בדמיונו השני לפי שיחס היתרון שבין ג' וד' הידוע אל היתרון שבין ד' והמספר הנעלם כיחס ג' הידוע אל ו' הנעלם והנה הנעלם גדול מיתרונו על ד' הנה אם כן ג' גדול יותר מהיתרון שבין ג' לד' מי"ד מה' אקלידיס | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והיתרון שבין ג' לד' ידוע אם כן כשחסרנוהו מג' יהיה הנשאר ידוע והוא ב' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וגם כן ידענו שד' הוא הנשאר כשחסרנו מו' היתרון שנוסף על ו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועתה יש לנו ג' מספרים ידועין | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
האחד ב' והוא הנשאר מג' כשחוסר ממנו היתרון שבין ג' לד' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
השני ג' שהוא הכל ידוע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
השלישי ד' שהוא הנשאר מהנעלם כשחוסר ממנו היתרון שהוא נוסף על ד' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וידענו שיחס המחוסר מג' אל המחוסר מהנעלם כיחס ג' שהוא הכל אל הנעלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם כן יחס ב' הנשאר אל ד' הנשאר כיחס ג' אל הנעלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולכן נכפול האמצעיים שהן ד' וג' והיו י"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונחלק י"ב על ב' והיה ו' וכו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Understand the others from Euclid's 18.V. | וכן תבין האחרים מי"ח מה' אקלידיס | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Proposition 16 is derived this way: the first and the seventh are taken, and they are cardinal points. One proposition is derived from both of them. From the fourth and tenth, which are cardinal points, another proposition is derived. Then, from both of them, one proposition is derived, called the 16th, and is the strongest of them all. | צורת הי"ו מוציאין אותה בדרך זו לוקחין הראשונה והשביעית והן יתדות ומוציאין משתיהן צורה אחת ומהרביעית והעשירית והן יתדות מוציאין צורה אחרת ומשתיהן מוציאין צורה אחת והיא נקראת הי"ו והיא חזקה מכלן | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| I have found, with the help of God, proposition 16: We take the first and seventh propositions and derive one proposition from both of them. We take proposition 15, and from these two, we derive another proposition, which is the 16th, and this is a secret. | ומצאתי בע"ה צורת הי"ו נקח הצורה הראשונה והשביעית ועושין משתיהן צורה אחת ונקח צורת הט"ו [87]ומשתים אלו נעשה צורה אחרת והיא הי"ו וזה סוד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Additional Word Problems (P1026) |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שאלה שלשה הלכו לקנות דג בשוק ערכו ה' פשו' אמר אחד אני אתן כל מה שבכיסי ואתם לא תתנו רק החצי שבכיסכם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נבקש המורה והוא י"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כ"ט כי ממנו נוכל לעשות ג' מספרים שישתוו שלשתן למספר אחד והן ה' י"א י"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
בעל ה' ישאל החצי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
בעל י"א ישאל השליש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
בעל י"ג ישאל הרביע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שאלה ראובן שמעון לוי ויהודה אמ' ראובן לשמעון כל מה שבכיסי עם חצי שבכיסך הוא ה' די' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תשובה ראובן ל"ז פשו' צ"ז חלקי' מקי"ו בפשו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שמעון מ"ד פשי' מ"ד חלקים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
לוי מ"ו פשו' ק"ו חלקים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
יהודה נ"ב פשי' נב חלקים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ובזה נמצא כל ראובן עם חצי שמעון ה' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן שמעון עם שליש לוי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן לוי עם רביע יהודה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן יהודה עם חומש ראובן | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נסיר חצי שמעון המשותף וישאר ראובן שוה לחצי שמעון עם שליש לוי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ושמעון שוה לשני שלישי לוי עם רביע יהודה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם כן ראובן שוה לשני שלישי לוי עם שמינית יהודה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם כן לוי שוה לג' רבעי יהודה עם חומש ראובן | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם כן ראובן שוה לה' שמיניות יהודה וב' חלקי' מט"ו מראובן עצמו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
א'פ'ד' ראובן ע"ה שמעון פ"ח לוי צ"ג יהודה ק"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
או ראובן ל"ז וחצי [88]שמעון מ"ד לוי מ"ו וחצי יהודה נ"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן לחציין וכן וכן | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Question: a man arrived to a country and took an oath that if he will double his money every day, he will would spend a certain amount of it each day. After a few days he had nothing left.
|
שאלה אדם נכנס [בע]יר ונודר שאם יכפול ממונו בכל יום יוציא ממנו בכל יום סך מוגבל ולימים לא נשאר בידו מאומה כמה הוא הממון שבידו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The method: we look for a number, such that, when doubled in a double ratio as many times as the number of days, at the end of which he has nothing left, the result is the amount he spends every day. We subtract this required number from the amount he spends each day and the remainder is the amount of money he has. | הדרך בזה שנבקש מספר שבהכפלו פעמים כמספר הימים על יחס הכפל שאחריהם לא נשאר לו מאומה יעלה לסך שיוציא מהם בכל יום והמספר ההוא המבוקש נגרע מהסך שיוציא בכל יום והנשאר הוא הממון שבידו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
המשל שיוציא בכל יום מ"ח פשי' ולסוף ג' ימים לא נשאר לו מאומה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה המספר שבהכפלו על יחס הכפל כמספר הימים שהם ג' יעלה מ"ח הוא ו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כי כשנכפול ו' יעלה י"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכשנכפול י"ב עלה כ"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכשנכפול כ"ד עלה מ"ח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נגרע מספר ו' ממ"ח וישאר מ"ב והם המעות שבידו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
או שיוציא בכל יום י"ח פשי' ולסוף ה' ימים לא נשאר לו מאומה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה המספר שבהכפלו על יחס הכפל פעמים כמספר הימים שהוא ה' יעלה י"ח הוא חצי וחלק אחד מי"ו שהן ט' חלקים מי"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כי כשנכפול חצי וחלק אחד מי"ו יעלה א' ושמינית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכשנכפול א' ושמינית יעלה ב' ורביע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכשנכפול ב' ורביע יעלה ד' וחצי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכשנכפול ד' וחצי יעלה ט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכשנכפול חמשית ט' יעלה י"ח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נגרע מספר חצי וחלק אחד מי"ו יעלה י"ז וז' חלקים מי"ו והם המעות שבידו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The reason the root is written in the middle rank, whether there is an odd number of ranks, or an even number, is that we shift the even rank above the preceding rank and add them together, as when we write 7 above the 9 and it becomes seventy. | [89]טעם למה יוציא השרש אל אמצע המדרגות בין שיהיו המדרגות נפרדים כמו זה או זוגות שנשים המדרגה הזוגיית למעלה מהמדרגה שלפניה שנחבר הכל כמו שנשים ז' למעלה מט' ויהיה שבעים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Since in every product, the ranks are doubled. After we square it, the product has six ranks. | לפי שלעולם בכל כפל המדרגות כפולות אחר שנעשה מהם מרובע מהכאתם עולה לששה מדרגות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If the result of multiplication has an even [number of ranks]: | אם יש ליוצא מההכאה זוגות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כמו זה שח' על ח' עולה לס"ד ולכן ס"ד הוא תחת המדרגה הששית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If the number [of the ranks] is odd, we take the root from [...] | ואם המספר נפרד לא נקח שרש כי אם מה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Notes
- ↑ דברי הימים ב, ב, טז
Apparatus
- ↑ P1054 31r
- ↑ P1054 om.
- ↑ 15r
- ↑ 15v
- ↑ 16r
- ↑ Guenzburg 193v
- ↑ MS Firenze: ביאור החלוק עשאו ה'ה'ר' עמנואל בן יעקב ע"ב בעל הכנפיים; MS London: כלל שעשה החכם הגדול ר עמנואל בן יעקב למצוא דרך קל לכל חשבון קראו חכם אמר; MS P1081 אמר עמנואל בן יעקב
- ↑ על מספרים רבים כמה שיהיו: MS P1081 om.
- ↑ בטור: MS L om.
הטור: MS L om.
וכו': Lומאיות תחת מאיות
שהטור: MS L וזה
הטור השפל: MS L טור שפל - ↑ הטור: MS L om.
- ↑ הם: Guenzburg: קח
- ↑ כמספר אחרון שבטור... לאחרון שבטור השפל: MS P1081 om.
- ↑ כשלשי לאחרון שבטור העליון לפניו: MS L om.
- ↑ מספר: MS L כמה
הפעמים: MS L פעמים
אשר: MS L om.
האמצעי: MS L אמצע
ותכתוב כמספר ההוא: MS L ותכתבינו
תקח: MS L יקח
שבטור: MS L om.
ממדרגת: MS L שבמדרגת - ↑ לך: MS L om.
לא: MS L om.
לקחת: MS L לדעת
פעם: MS L om.
המספר: MS L מספר
האחרון: MS P1081 אחרון
שבטור: MS P1081 שבטור שבטור
הוא: MS L om.
הוא א' ... העליון: MS P1081 om.
הוא: MS L om. - ↑ כל המספר ... ר"ל: MS L om.
הח': MS L ח'
יהיו: MS L יהיה
ותראה: MS L וראה
יהיה: MS P1081 הוא
Guenzburg: בפניו
בעצמך: MS L כל כך
המספר: MS L מספרו
בפ"ט: Guenzburg om.
שבטור העליון: MS L שבטור הח'
ט': MS L om.
ועם: MS Lחסרינועם
הנה: MS L om.
כן: MS L om.
ד' במספר פ"ד: MS L om. - ↑ ט': MS L ד' ט'
ממדרגתו: MS L ממדרגות
בט': MS P1081 הד'
במדרגת: MS L במדרגות - ↑ Guenzburg 194r
- ↑ כן: MS L om.
וכפול: MS L אחר זה כפול
האות: Guenzburg om.
זאת האות שיצא לך: MS L אשר יצא
L: הכפל ר"ל ט' על ב' על ג' על ד' על ט'
מהטור: MS L מטור
או מן האחדים: MS L om.
אחר: MS L מן
הטור: MS L טור - ↑ שוב: MS L ישוב
על הדרך ... הטור השפל: MS L om.
מהכפל: MS L om.
מן הנשאר: MS L מהנשאר - ↑ ואחר: MS L אחר כן
ולכפול: MS L לכפול - ↑ לזה הדרך: MS L om.
- ↑ בטור: MS P1081 הטור
- ↑ שבטור: MS L שהוא בטור
- ↑ עשית: MS L עשיתה
העליון: MS P1081 עליון
שבטור: MS L שנשמר בטור
נעשית מלאכתך: MS L מלאכתך נעשית
בזולת: MS L מבלי
בה: MS L om.
תחבר: MS P1081 תסדר
יצאו: Guenzburg שאו
כל: MS L om.
וגם: MS L גם
מנהג: MS L om.
ידעת מנהגו: MS L om.
העליון: MS P1081 עליון
הנה: MS L om.
החשבון: MS L חשבונך
שום התחלפות: MS L om.
הנה: MS L om. - ↑ L: אמר החכם הנז'
- ↑ לך: L om.
מה שיש בו: L om.
מן ההזכרה: L מההזכרה
היותר: P1081 margin
להם: MS L om.
אשר אין: MS L שאין
שלמים: MS L שברים
נשברים: MS L השברים
או: MS L הן
לבדם: MS L om.
מן ההזכרה: MS L מהזכרה - ↑ ומעתה: MS L ומזה
- ↑ במעלת: MS L במעלות
בה: MS P1081 בו
וארבעה: MS L ארבעה - ↑ ובמעלה: MS P1081 והמעלה
השנית: MS L שינית
שהיא מעלת עשרות: MS L om.
בה: MS L בו
כלל: MS L om.
עם: MS L om.
ולא: MS L לא
MS L: אין בהם שום מספר מרובע
אמנם: MS L רק
אחדים: MS L האחדים
בה: MS L בו
ר"ל: Guenzburg ול"י
שנוסיף על מספר: MS L שנשיב אחור
אחדים מה: MS L ונחברם עם אחדים
שרשו: MS L ששורשו five times - ↑ מעלת: P1081: מעלות; L: מעלה ממעלות
ודומיהן: MS L וכן
למעלה: MS L למעלו' - ↑ במעלת: MS L במעלות
המאיות: MS Lהאחדי'המאיות
מדרגת: MS L om.
גם כן: MS L om. - ↑ שדומה: Guenzburg ששרשו
- ↑ וארבע: MS L ד'
אחדים: MS L om. - ↑ וט': MS L ט'
אחדים: MS L om.
לשלש: Guenzburg לשלש הם; MS L: וכן כל מעלה שמספר מדרגתה נפרד כמו חמישי' ושביעי' ותשיעי' וכן לאין קץ - ↑ וכן כל: MS L וכל
שמספר: MS L om.
והיא: MS P1081 והוא
מעלה: MS L מעלת
זוגיית: MS L זוגות
בה מרובעים ... יש בה: MS L om.
המאיות: MS Lעשרותמאיות
שנשים: MS L שנשיב
ואלף: MS L אלף
ותחבר: MS L ונסדר
עם: MS L כל - ↑ Guenzburg 194v
- ↑ עשרה: MS L עשר
לארבעה: MS L לארבע - ↑ לל"ו: MS P1081 ל"ו
ששים הדומה: MS L om.
לששה: MS L ו' - ↑ וח' אלפים ומאה: MS P1081 ומאה אלפים ושמנה
- ↑ וכן: Guenzburg וכל
שמינית: Guenzburg שמנים - ↑ במעלת: Guenzburg במעלת במעלת; MS P1081 המעלה
האמצעית: MS P1081 הממוצעת - ↑ מספר: MS L om.
הענין: MS L om. - ↑ ושרשו: MS L שרשו
ב': Guenzburg om.
הרביעית: MS L רביעית כמו העניין בצורה; Guenzburg om.
המעלה: MS L למעלה - ↑ כמו שבארנו: MS P1081 om.
אחד: MS P1081 om.
שבמעלה: Guenzburgשמשבמעלה
ואז: MS P1081 ואם
ישובו: MS P1081 יש בו - ↑ האחרונה: MS L
הראשונההאחרונה
אשר ילקח: MS L שילקח
בטור: MS P1081 ביאור - ↑ אשר לוקח: MS L שלוקח
ר"ל שזה וזה: MS L נ'
מן האחדים: MS L מהאחדים - ↑ זה: MS L om.
אזהירך: MS L אזכירך
להזכירך: MS L להזהירך
במדרגת: MS P1081 במדרגות
א': MS L ב'
ד': MS P1081 א'
פ"א: MS P1081 פ"א פ"א - ↑ כי המעלה הנפרדת הנה: MS L om.
- ↑ בידיעת: MS P1081 וידיעת
שרש: MS P1081 השורש
להם: MS L לו - ↑ אשר תרצה: MS L שתרצה
- ↑ א' ... ה': MS L om.
- ↑ ה': MS L om.
הוא: MS L om.
ה': MS P1081 om.
לו: MS L om.
שורש: MS L om. - ↑ הג': MS L ג'
ונרשום: MS L וירשום
ריוח: MS L om.
אות השרש: MS L האותיות - ↑ 195r
- ↑ ממנה: MS L om.
ולכתוב ... מן האחדים: MS L om.
שני: MS P1081 שבו
מדרגות: MS L המדרגות
התחלת: MS L התחלה
מהמספר: MS L המספר
במינו: MS P10181 ממינו
ישאר: MS L יהיה - ↑ ג': MS P10181 om.
תכתוב: MS L תכפול
תחתיה: MS L תחתיו
ואם: MS Lוהאםואם
שהיה: MS L שאם היה
השרש הכפול: MS L שורש הכפל
בשנים: MS L om. - ↑ מהמספר הראשון: MS L om.
זאת: MS L אות
תאמר: MS P1081 אומר
ונשיבהו: MS L והשיבו העם הד'
נוכל: MS L תוכל
לקחת: Guenzburg om.
המספר: MS L om. - ↑ זאת הג': MS L ג'
ואחר על: MS L ועל - ↑ ונתחיל: MS L ותתחיל
הנופלת: MS P1081 הכופלת - ↑ מן הנשאר: MS L מהנשאר
במינו: MS L מן מינו
העליון: MS P1081 עליון - ↑ זאת: MS L om.
זאת: MS P1081 זה
השנית: MS P1081 השני
מן השורש: MS L מהשורש
שהיא: MS L שהוא - ↑ באופן שנוכל: MS P1081 om.
המספר: MS L om.
מן החלוקה: MS L מהחלוקה
והיא: MS P1081 והוא - ↑ ונכפול זאת הג' ... היא במעלת האחדים: MS P1081 om.
- ↑ למעלה: MS L om.
- ↑ אמנם: MS L om.
- ↑ 195v
- ↑ האחרון: MS L הראשון
גם כן: MS L ג"כ
אשר תקח: MS L שתקח
השרש: MS L ראש השורש
אשר לפני: MS L שלפני - ↑ תשוב: MS P1081 תשיב
כפול: MS L כפלו
אשר לקחת: MS L שלקחת
ממנו: MS P1081 om.
מהמספר: MS L ממספר
אשר אחריו: MS L שאחריו
תראה כי: MS L om.
השורש: MS L השורשהקרוב והואא
כל: MS L om.
כמו שנראה: MS L om. - ↑ רצינו ... ט'ט'ג': MS L om.
- ↑ רצינו ... לקחנו: Guenzburg twice
מהמספר: MS L ממספר - ↑ והיא: MS L והוא
- ↑ הנשאר: MS L ב' הנשאר
מהנשאר: MS P1081 מהראשון מהנשאר
מפני כי: MS L ומפני זה
יהיה: MS L om.
אשר לקחנו: MS L שלקחנו
הא': MS L הג'
ראשונה: MS L ראשון
נכתב: MS P1081 ב' נכתב
כמשפט: MS L כמספר
אשר לפניה: MS L שלפניה
כ"ט: MS L om. - ↑ המספר: MS L מספר
במחולק: MS P1081 במחלק
יהיה: MS P1081 נקוה
כי: MS L om.
ואף כי יותר מעשרה: MS L om.
זה: MS L om. - ↑ נשוב: MS P1081 נשאר
כשכפלנו: MS P1081 השפלנו
הוספנו: MS L נוסף
והנה: MS L הנה
לא הוספנו עליו... כשכפלנו זה השורש: Guenzburg om.
כבר: MS L om.
נתוספה... ומאה הנה: Guenzburg om.
ותוספת: MS P1081 נוספה
לחלק: MS L om.
גם כן: MS L ג"כ
הכפול: MS L כפול
מן האחדים: MS L מהאחדים - ↑ P903 138v
- ↑ Guenzburg 196r
- ↑ Guenzburg 196v
- ↑ Guenzburg om.
- ↑ שעבר: Guenzburg שנים
- ↑ 76r
- ↑ 76v
- ↑ 77r
- ↑ 77v
- ↑ 78r
- ↑ 78v
- ↑ 79r
- ↑ 79v
- ↑ 80r
Appendix: Bibliography
Immanuel ben Jacob Tov-Elem / Immanuel Bonfils / Immanuel of Trascon (flourished c. 1340-1377)
Manuscripts:
- 1) Firenze, Biblioteca Medicea Laurenziana Plut. 88.30/2 (IMHM: f 17853), ff. 37r-38r (15th century)
- Plut. 88.30/2
- 2) London, British Library Or. 10878 (IMHM: f 8193), ff. 6r-7v (15th century)
- Or. 10878
- 3) Moscow, Russian State Library, Ms. Guenzburg 365/11 (IMHM: f 43035), ff. 193v-196v (15th-16th century)
- Guenzburg 365
- 4) Paris, Bibliothèque Nationale de France heb. 903/7 (IMHM: f 26859), ff. 138r-140r (15th century)
- heb. 903/7
- 5) Paris, Bibliothèque Nationale de France heb. 1026/6 (IMHM: f 15025), ff. 72r-80v (16th century)
- heb. 1026/6
- 6) Paris, Bibliothèque Nationale de France heb. 1054/8 (IMHM: f 33997), ff. 31r-v (15th century)
- heb. 1054/8
- 7) Paris, Bibliothèque Nationale de France heb. 1081/3 (IMHM: f 15037), ff. 4r-15v (16th-17th century)
- heb. 1081/3
Bibliography:
- Gandz, Solomon. 1936. The Invention of the Decimal Fractions and the Application of the Exponential Calculus by Immanuel Bonfils of Tarascon (c. 1350), Isis XXIV, pp. 16–45.
- Lévy, Tony. 2003. Immanuel ben Jacob de Tarascon (XIVe s.): fractions décimales, puissances de 10 et opérations arithmétiques, Centaurus 45, pp. 284-304.
- ———. 2012. Immanuel ben Jacob of Tarascon (Fourteenth Century) and Archimedean Geometry: An Alternative Proof for the Area of a Circle, Aleph. 12.1, pp. 135-159.
- Rabinovitch, Nahum. 1974. An Archimedean Tract of Immanuel Tov-Elem (14th Cent.), Historia Mathematica 1, pp. 13–27.
- Rashed, Roshdi. 1994. The development of Arabic mathematics: between arithmetic and algebra. Translated by A.F.W. Armstrong. Dordrecht, Boston, London: Kluwer Academic Publishers, pp. 85-146.
- Sarton, George. 1934. Simon Stevin of Bruges (1548-1620), Isis, vol. 21, no. 2 (Jul., 1934), pp. 241-303.
- ———.1935. The First Explanation of Decimal Fractions and Measures (1585). Together with a History of the Decimal Idea and a Facsimile (No. XVII) of Stevin's Disme, Isis, vol. 23, no. 1 (Jun., 1935), pp. 153-244.
- Steinschneider, Moritz. 1893-1901. Mathematik bei den Juden. Berlin-Leipzig-Frankfurt: Kaufmann, pp. 155-163 (f79-f87); repr. Hildesheim: G. Olms, 1964 and 2001.

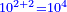
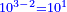
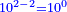
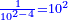



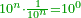
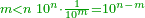


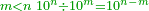

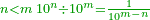
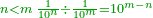
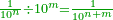

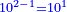
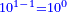

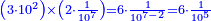
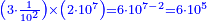
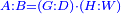
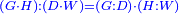
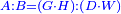

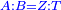

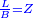

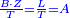
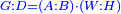
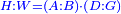
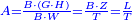
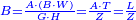

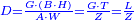
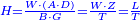
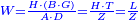
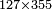
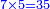



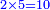
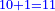

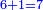
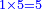



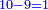

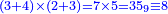
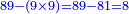
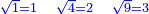


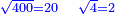
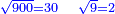


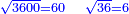
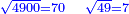
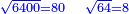
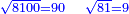

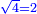
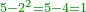



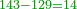

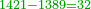

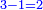


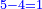

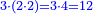
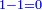


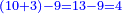

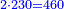
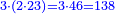
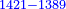


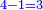
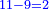
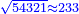
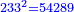
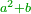
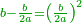
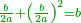
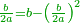
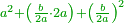
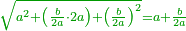
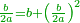

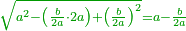
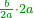
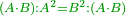
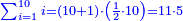
![\scriptstyle{\color{blue}{\sum_{i=1}^7 i=\left[\frac{1}{2}\sdot\left(7+1\right)\right]\sdot7=\left(\frac{1}{2}\sdot8\right)\sdot7=4\sdot7}}](/mediawiki/images/math/2/f/9/2f9d158f8016c8cb4b4682a2f2ba2880.png)
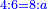
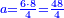
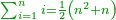


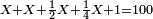
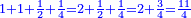
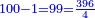
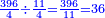
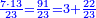
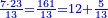
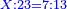
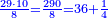
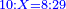
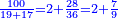
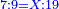
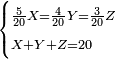
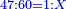
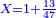


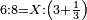
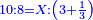
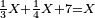
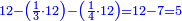
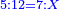
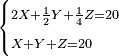
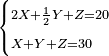
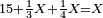
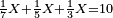
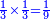
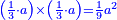
![\scriptstyle{\color{blue}{10\sdot\left[\left(\frac{1}{3}\sdot a\right)\times\left(\frac{1}{3}\sdot a\right)\right]-\left[\left(\frac{1}{3}\sdot a\right)\times\left(\frac{1}{3}\sdot a\right)\right]=\left(10\sdot\frac{1}{9}a^2\right)-\frac{1}{9}a^2=a^2}}](/mediawiki/images/math/1/5/3/1539bb9d90b89d1bbdf434c812799c7e.png)

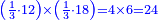
![\scriptstyle{\color{blue}{12\times18=10\sdot\left[\left(\frac{1}{3}\sdot12\right)\times\left(\frac{1}{3}\sdot18\right)\right]-\left[\left(\frac{1}{3}\sdot12\right)\times\left(\frac{1}{3}\sdot18\right)\right]=10\sdot\left(4\times6\right)-\left(4\times6\right)=240-24=216}}](/mediawiki/images/math/d/4/4/d449de85c5f08ba1ff02e8b408675e17.png)


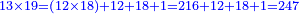

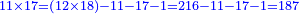





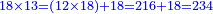
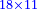
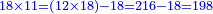

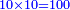

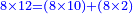
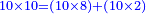
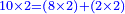
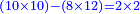
![\scriptstyle{\color{OliveGreen}{a\times b=\left[a+\frac{1}{2}\sdot\left(b-a\right)\right]^2-\left[\frac{1}{2}\left(b-a\right)\right]^2}}](/mediawiki/images/math/e/d/0/ed01f0b5b97ba1e273ab440c8b165242.png)

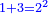
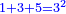

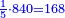

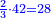
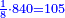
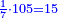
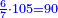
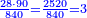
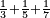
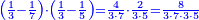


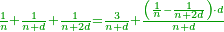
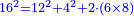

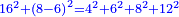
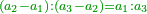


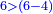
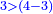
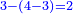
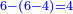
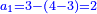
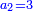
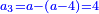

![\scriptstyle{\color{blue}{\left[3-\left(4-3\right)\right]:\left[a-\left(a-4\right)\right]=2:4=3:a}}](/mediawiki/images/math/f/5/d/f5da44eab4e77077a8ed83f8b0f949aa.png)