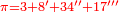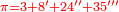Difference between revisions of "ספר המספר / אברהם אבן עזרא"
(→Numeration) |
(→Chapter Three – Addition) |
||
| (156 intermediate revisions by the same user not shown) | |||
| Line 5: | Line 5: | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|ראה ספר מחוקק באמונה | + | |style="text-align:right;"|ראה ספר מחוקק באמונה |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|ותמצא בו | + | |style="text-align:right;"|ותמצא בו לכל מספר תכונה |
|- | |- | ||
| | | | ||
| Line 14: | Line 14: | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|קטן שנים וחכם בתבונה‫<ref>ראה ... בתבונה: B עמ"י עש"ו בהנו"א; Lo27153 בשם השם אמן עמ"י עש"ו; Lo10785 בהנו"א עמ"י עש"ו; V397 ע"ז; Mu43; O187; P1029; P1050; P1051; W152; W194 om.</ref> | + | |style="text-align:right;"|קטן שנים וחכם בתבונה‫<ref>באמונה: Mu150 ב<sup>א</sup>מונה<br> |
| + | בו: Mu150 top<br> | ||
| + | ראה ... בתבונה: B עמ"י עש"ו בהנו"א; Lo27153 בשם השם אמן עמ"י עש"ו; Lo10785 בהנו"א עמ"י עש"ו; V397 ע"ז; Mu43; O187; P1029; P1050; P1051; W152; W194 om.</ref> | ||
|- | |- | ||
| | | | ||
| − | + | |style="text-align:right;"|<big>ספר המספר</big>‫<ref>ספר המספר: B ס' יסוד מספר; Lo27153 אתחיל לכתוב ספר המספר מאע"ז ז"ל; Mu43 לק"י אתחיל ספר המספר לר' אברהם אבן עזרא ז"ל; Lo10785 ספר המספר להחכם ר"א ן' עזרא; O187 מספר לר' אברהם בן עזרא; P1029 מספר ר' אברהם ן' עז'; P1050 ספר המספר להחכם ר' אברהם אבן עזרא נ"ע; V397 ספר המספר שחבר החכם ר' אברהם ן' עזרא ז"ל; W152 ספר המספר לא"ע נ"ע; Mu150; P1051; V171; V386; W194 om.<br> | |
ראה ... המספר: Mo30 עמי עשו<br> | ראה ... המספר: Mo30 עמי עשו<br> | ||
ספר המספר לכ' אברהם ב"ע ז"ל<br> | ספר המספר לכ' אברהם ב"ע ז"ל<br> | ||
| Line 44: | Line 46: | ||
|- | |- | ||
|Since the sublime God alone has created in the upper world nine large heavenly spheres revolving around the earth, which is the lower world. | |Since the sublime God alone has created in the upper world nine large heavenly spheres revolving around the earth, which is the lower world. | ||
| − | |style="width:45%;text-align:right;"| | + | |style="width:45%;text-align:right;"|<div id="Since God"></div>בעבור‫<ref group=note>[[Excerpts_Attributed_to_Immanuel_Bonfils#Since|<span style=color:blue>commentary: since</span>]]</ref> כי השם הנשגב לבדו ברא ב{{#annot:upper world|2075|7Vq1}}עולם העליון{{#annotend:7Vq1}} תשע {{#annot:heavenly sphere|2075|dnD1}}עגולות{{#annotend:dnD1}} גדולות {{#annot:term|274,2211|gOMD}}סובבות את{{#annotend:gOMD}} {{#annot:earth|2075|hJTk}}הארץ{{#annotend:hJTk}} שהוא {{#annot:sublunar world|2075|Vk6W}}העולם השפל{{#annotend:Vk6W}}‫<ref>בעבור: Mu43 אמר בעבור<br> |
| + | הנשגב: O187 om.; V171 הנה‫'<br> | ||
| + | לבדו: Lo10785 לבדו ב"ה; N2627; V171 om.<br> | ||
| + | בעולם: Mu43; O187; V171; W152; W194 העולם<br> | ||
| + | העליון: P1050 om.<br> | ||
| + | גדולות: P1029; P1051 om.<br> | ||
| + | את: B top; V171; V397 om.<br> | ||
| + | שהוא: Lo27153; P1050; W194 שהיא; B שהוא marg. נ' שהיא<br> | ||
| + | שהוא העולם: V171 שהעולם</ref> | ||
|- | |- | ||
|<span style=color:green>Reference to the triple repetition of the word sefer in Sefer Yezira [the Book of Creation] – interpretation of one of them as representing the concept of the nine numbers:</span> | |<span style=color:green>Reference to the triple repetition of the word sefer in Sefer Yezira [the Book of Creation] – interpretation of one of them as representing the concept of the nine numbers:</span> | ||
| Line 50: | Line 60: | ||
|- | |- | ||
|And the author of the Sefer Yezira has said that the paths of the wisdom are by Sefar and Sefer and Sipur and the Sefar is nine numbers. | |And the author of the Sefer Yezira has said that the paths of the wisdom are by Sefar and Sefer and Sipur and the Sefar is nine numbers. | ||
| − | |style="text-align:right;"|<div id="author of the Sefer Yezira"></div>ובעל ספר<ref> | + | |style="text-align:right;"|<div id="author of the Sefer Yezira"></div>ובעל ספר יצירה‫<ref group=note>[[Excerpts_Attributed_to_Immanuel_Bonfils#The_author_of_the_Sefer_Yezira|<span style=color:blue>commentary: The author of the Sefer Yezira</span>]]</ref> אמר כי {{#annot:term|277|FVUG}}נתיבות החכמה{{#annotend:FVUG}} הם <div id="Sefar"></div>בִּסְפָר‫<ref group=note>[[Excerpts_Attributed_to_Immanuel_Bonfils#Sefar|<span style=color:blue>commentary: Sefar</span>]]<br> |
| − | {{#annot:definition|287|tNZb}}והנה הַסְּפָר<ref>הַסְּפָר: Mu43 הַסְפַר; N2627; P1050 המספר; P1052 הספור; V171 הסופר< | + | Mo marg. פי' ספר כתב הלשון ספר הדבור; P1052 top במשמע' שפה</ref> <div id="Sefer"></div>וְסֵפֶר‫<ref group=note>[[Excerpts_Attributed_to_Immanuel_Bonfils#Sefer|<span style=color:blue>commentary: Sefer</span>]]</ref> <div id="Sipur"></div>וְסִפּוּר‫<ref group=note>[[Excerpts_Attributed_to_Immanuel_Bonfils#Sipur|<span style=color:blue>commentary: Sipur</span>]]<br> |
| + | וְסִפּוּר: P1052 top מניי‫'<br> | ||
| + | סְפָר וְסֵפֶר וְסִפּוּר: B com ביאור ההקדמה הנזכרת. סְפָר וְסֵפֶר וְסִפּוּר פי' כי הדבור אצל ציור הלב והרעיון בצורה הנראת כמראה אצל עצם הדבר אשר היא צורה לו כי הציורים הם בשכלו נמצאים והציור הוא צורת עצם הדבר והדבור חקוי הציור והמכתב חקוי הדבור והם ספר וספר וספור. ובעל ספר יצירה לא זכר מציאות העצם שהוא העקר והקודם אבל השלשה הנמצאי' בנתיבות החכמה כך פי' אדונינו מאור הגולה החבר הידוע ר' משה בר' שמואל בן תבון ז"ל</ref><br> | ||
| + | {{#annot:definition|287|tNZb}}והנה הַסְּפָר תשעה {{#annot:term|204|1E4G}}מספרים{{#annotend:1E4G}}‫<ref>ובעל ספר: O187 וספר<br> | ||
| + | ספר יצירה: V171 ס"י<br> | ||
| + | אמר: O187 אומר<br> | ||
| + | החכמה: O187; P1029; P1051; V171; V397 חכמה<br> | ||
| + | וספר: P1051 om.<br> | ||
| + | בִּסְפָר וְסֵפֶר וְסִפּוּר: Lo10785; Mo30 בסֶפֵר וסְפַר וְסיפור<br> | ||
| + | הַסְּפָר: Mu43 הַסְפַר; N2627; P1050 המספר; P1052 הספור; V171 הסופר<br> | ||
| + | תשעה: V171; V397 הם ט‫'</ref> | ||
|- | |- | ||
| | | | ||
| Line 60: | Line 80: | ||
| | | | ||
::For nine is the end of every number and these are called units that are the first rank. | ::For nine is the end of every number and these are called units that are the first rank. | ||
| − | |style="text-align:right;"|כי תשעה | + | |style="text-align:right;"|כי תשעה סוף כל {{#annot:term|204|z6R9}}חשבון{{#annotend:z6R9}} ואלה יקראו {{#annot:term|287|f4NJ}}האחדים{{#annotend:f4NJ}} שהם ב{{#annot:term|203|dJ7O}}מעלה{{#annotend:dJ7O}} הראשונה{{#annotend:tNZb}}‫<ref>חשבון: P1052 מספר<br> |
| + | ואלה: P1052 ואלו<br> | ||
| + | יקראו: P1029 יקרא<br> | ||
| + | האחדים: Lo10785; Mo30 כאחדים; Mu43; N2627; P1029; V397 אחדים<br> | ||
| + | הראשונה: N2627; P1029; V397; W152; W194 ראשונה; P1052 הראשו<s>ש</s>נה הראשונה marg. יקראו האחדי' שה' במע' הרא' היה הנקודה<br> | ||
| + | תשעה: Lo10785; Mo30 הט‫'</ref> | ||
|- | |- | ||
| | | | ||
| Line 68: | Line 93: | ||
| | | | ||
::Because ten resembles to one and twenty resembles to two. | ::Because ten resembles to one and twenty resembles to two. | ||
| − | |style="text-align:right;"|כי עשרה | + | |style="text-align:right;"|כי עשרה {{#annot:term|2506|z6f9}}דומה ל{{#annotend:z6f9}}אחד<br> |
| − | ועשרים דומים<ref>דומים: B; Mo30 (marg.); Mu150; P1051; V171; V397 דומה< | + | ועשרים דומים לשנים שהם שני עשרות‫<ref>עשרה: Mu43; N2627; P1029; P1051 העשרה; V171 היוד; V386 יוד<br> |
| + | דומים: B; Mo30 (marg.); Mu150; P1051; V171; V397 דומה<br> | ||
| + | לאחד ... לשנים: Lo10785 ל<br> | ||
| + | שני: P1050 שתי</ref> | ||
|- | |- | ||
| | | | ||
| Line 77: | Line 105: | ||
| | | | ||
::It would be appropriate to call it "‛esrayim", as "matayim" [= two hundred] is called from "meah" [= one hundred] and "alpayim" [= two thousand] from "eleph" [one thousand], but for the following numbers that are šelošim [thirty] to tiš‛im [ninty] it has been treated in their usage. | ::It would be appropriate to call it "‛esrayim", as "matayim" [= two hundred] is called from "meah" [= one hundred] and "alpayim" [= two thousand] from "eleph" [one thousand], but for the following numbers that are šelošim [thirty] to tiš‛im [ninty] it has been treated in their usage. | ||
| − | |style="text-align:right;"|והיה<ref>והיה: P1051 יהיה; V171 שיהיה< | + | |style="text-align:right;"|והיה ראוי שיקראוהו עֶשְׂרַיִם כאשר יקראו ממאה מאתים ומאלף אַלְפַּים<br> |
| − | + | רק בעבור חבריו {{#annot:term|404|hWcF}}הבאים אחריו{{#annotend:hWcF}} שהם שלשים עד תשעים נהגו כמנהגם‫<ref>והיה: P1051 יהיה; V171 שיהיה<br> | |
| + | שיקראוהו: Lo27153 <s>שיקרואו</s> שיקראוהו; Mu43; P1051 שיקראהו; O187 לקרות; P1050; P1052 שיקראו<br> | ||
| + | עֶשְׂרַיִם: Mu43 עשָרים; O187 עַשַרַיִם; P1052 עֲשָרָיִים; B עֲשַרָיִם marg. נ' עֱשְרַיִם<br> | ||
| + | יקראו: O187; V171 קראו<br> | ||
| + | ממאה: V171 מהמאה<br> | ||
| + | ומאלף: V171 ומהאלף<br> | ||
| + | רק בעבור: Lo10785 om.<br> | ||
| + | אחריו: Mu150 לפניו marg. אחריו<br> | ||
| + | שהם: Lo10785 om.; V171 שלהם<br> | ||
| + | הבאים אחריו שהם: P1051 om.<br> | ||
| + | שלשים: V397 משלשים<br> | ||
| + | נהגו: B; O187 נהגוהו; V171 יהגהו<br> | ||
| + | נהגו כמנהגם: Lo10785 נהגו ר' נהגם</ref> | ||
|- | |- | ||
| | | | ||
::So šelošim is derived from šaloš [= three] and so all of them. | ::So šelošim is derived from šaloš [= three] and so all of them. | ||
| − | |style="text-align:right;"|והנה שלשים | + | |style="text-align:right;"|והנה שלשים מ{{#annot:derivation|295|JfzZ}}גזרת{{#annotend:JfzZ}} שלש וככה כלם‫<ref>שלשים: P1052 om.<br> |
| + | שלש: O187 שלש וארבעים מגזרת ארבע<br> | ||
| + | כלם: Lo10785 om.</ref> | ||
|- | |- | ||
| | | | ||
:*<span style=color:green>hundreds and their resemblance to the units</span> | :*<span style=color:green>hundreds and their resemblance to the units</span> | ||
::One hundred resembles to one as well as to ten; two hundred resembles to two as well as to twenty. | ::One hundred resembles to one as well as to ten; two hundred resembles to two as well as to twenty. | ||
| − | |style="text-align:right;"|והנה מאה<ref>מאה: III המאה< | + | |style="text-align:right;"|והנה מאה דומה לאחד גם לעשרה<br> |
| − | + | ומאתים דומה לשנים גם לעשרים‫<ref>מאה: III המאה<br> | |
| + | דומה: P1050 דומים; W194 הדומה<br> | ||
| + | גם: III; Mu43 וגם<br> | ||
| + | לעשרה: P1029; P1050 עשרה<br> | ||
| + | ומאתים: Mu43 ומתים<br> | ||
| + | דומה: P1052 דומים; P1051 om.; B דומה marg. נ' דומים<br> | ||
| + | לשנים ... לעשרים: B; Lo27153(marg.); O187; P1051; V171 לעשרים גם לשנים; Mu43 לעשרים וגם לשנים<br> | ||
| + | דומה ... לעשרים: Lo10785; Mo30; Mu150; P1050; V386; V397 דומים לעשרים גם לשנים; W152 דומה לעשרים; W194 marg. דומים לעשרי‫'</ref> | ||
|- | |- | ||
| | | | ||
:*<span style=color:green>thousand and upwards</span> | :*<span style=color:green>thousand and upwards</span> | ||
::likewise the thousand and the ten thousand, because they are the beginning of decades of the numbers that follow them, which are 1, 10, 100; 2, 20, 200. | ::likewise the thousand and the ten thousand, because they are the beginning of decades of the numbers that follow them, which are 1, 10, 100; 2, 20, 200. | ||
| − | |style="text-align:right;"|וככה אלף ורבבה שהם ראשי | + | |style="text-align:right;"|וככה אלף ורבבה שהם ראשי {{#annot:term|299|cKsd}}כללים{{#annotend:cKsd}} למספרים הבאים אחריהם שהם א'י'ק' ב'כ'ר‫'<ref group=note>P1052 marg. איק בכר גלש הא' מן איק הוא ראשו' ל[אחדים] והיו' הו' ראשו' לעשריו' והקוף הו' ראשו' למאו'</ref><ref>ראשי: P1052 ראשים<br> |
| + | למספרים: Mu150 <sup>ל</sup>מספרים<br> | ||
| + | כללים למספרים: V171 מספרים כלליים<br> | ||
| + | אחריהם: O187 om.<br> | ||
| + | שהם: Mu43 שמהם<br> | ||
| + | א'י'ק' ב'כ'ר‫': Lo10785, Mo30; Mu150; N2627 (adds in marg.); V386 איק בכר גלש דמת הנך וסם זען חפף טצץ; W152 א'ק‫' [marg. א'י'ק'] ב'כ'ר' ג'ל'ש' ד'מ'ת וכו‫'; Mu43; O187; P1052; W194 א'י'ק' ב'כ'ר' ג'ל'ש‫'; P1029 אי"ק בכ"ר גל"ש דמ"ת</ref> | ||
|- | |- | ||
| | | | ||
| Line 101: | Line 155: | ||
| | | | ||
:The sign for this: when you draw a circle and write nine digits around it. | :The sign for this: when you draw a circle and write nine digits around it. | ||
| − | |style="text-align:right;"|ו{{#annot:term|198,1332|sGKK}}האות{{#annotend:sGKK}} על זה | + | |style="text-align:right;"|<div id="the_sign_for_this"></div>ו{{#annot:term|198,1332|sGKK}}האות{{#annotend:sGKK}} על זה‫<ref group=note>והאות על זה: B marg. ר"ל היות כל מספר סובב על ט'; B com. האות על זה. ר"ל האות על היות כל מספר סובב על ט'; W194 marg. כלומר על היות כל מספר סובב<br>Mu43 פי' והאות על היות כל מספר סובב על ט' והוא תכלית כל מספר כי כש[תכפ]ול ט' על כל המספרים שמא' עד ט' לעולם המחובר ט' לפי מספר האותות הצורה זל וזה א'וח' וזה ב"ז ג"ז ד‫'</ref>‫<ref group=note>[[פירוש_אנונימי_לספר_המספר#The_sign_for_this|<span style=color:blue>commentary: the sign for this</span>]]</ref> כשתעשה {{#annot:term|304|oaVK}}עגול{{#annotend:oaVK}}‫<ref group=note>כשתעשה עגול: B. com כשתעשה עגול וכו'. וכן היה יכול ליתן הבדל אחר כי כשתכפול ט' על עצמו או על ח' או על ז' או על ו' תמצא האחדי' שבמקו' העשרות יתרים במספרם מן האחדי' עצמם. ומה' ולמעלה וה' בכלל הדבר בהפך ר"ל שהאחדים עצמם יתרים על העשרות וזה כי ט' על ט' הם פ"א הנה ח' שהוא עשרות יתר על א'. וט' על ח' ע"ב הנה ע' שהם עשרות יתרי' על ב' שהם אחדים. ומהחמשה ולמטה יהיו האחדים עודפי' על העשרות</ref> ותכתוב סביבו תשעה מספרים‫<ref>והאות על זה: W152 כלומר על היות כל מספר סובב והאות על זה<br> |
| + | כשתעשה: Lo10785; Mo30 כי כשתעשה<br> | ||
| + | עגול: W152 top<br> | ||
| + | סביבו: O187 סביבותיו</ref> | ||
|- | |- | ||
| | | | ||
| Line 108: | Line 165: | ||
| | | | ||
:You multiply nine by itself; the reason is its being a square whose length is as its width; see it and so it is: | :You multiply nine by itself; the reason is its being a square whose length is as its width; see it and so it is: | ||
| − | |style="text-align:right;"|ותכפול תשעה | + | |style="text-align:right;"|ותכפול תשעה על עצמו וה{{#annot:term|311|VD8G}}טעם{{#annotend:VD8G}} להיותו {{#annot:term|305|IbQn}}מרובע {{#annot:term|316|tTbn}}ארכו{{#annotend:tTbn}} כ{{#annot:term|317|FXNE}}רחבו{{#annotend:FXNE}}{{#annotend:IbQn}} תראה זה וככה הוא‫<ref>תשעה: P1051 התשעה<br> |
| + | תשעה על: Mu43 שעל<br> | ||
| + | והטעם: Lo10785 ומטעם<br> | ||
| + | להיותו: Mu43; N2627; O187; P1029 להיות<br> | ||
| + | תראה זה: P1052; V386 om.<br> | ||
| + | תראה ... הוא: Lo10785; Mo30; Mu150 שהוא ט' פעמים ט‫'; P1050 om.</ref> | ||
|- | |- | ||
| | | | ||
::*The square is eighty-one, so the one is on the left of the nine, which is the beginning of the units; and the 8, which corresponds to eighty in the decade, is on the right of the nine. | ::*The square is eighty-one, so the one is on the left of the nine, which is the beginning of the units; and the 8, which corresponds to eighty in the decade, is on the right of the nine. | ||
:::<math>\scriptstyle9\times9={\color{blue}{8}}{\color{green}{1}}</math> | :::<math>\scriptstyle9\times9={\color{blue}{8}}{\color{green}{1}}</math> | ||
| − | |style="text-align:right;"|והנה המרובע<ref>המרובע: Lo10785; Mo30; O187; V397 מרובע ט'; Mu150; P1029; V386 המרובע מהט'< | + | |style="text-align:right;"|והנה המרובע אחד ושמונים והנה האחד לשמאלו של תשעה שהוא ראש האחדים וח' שהוא כנגד שמונים בכלל לימין תשעה‫<ref>המרובע: Lo10785; Mo30; O187; V397 מרובע ט‫'; Mu150; P1029; V386 המרובע מהט‫'<br> |
| + | אחד ושמונים: B; Lo10785; Mo30; O187; P1029; V171 פ"א<br> | ||
| + | והנה: N2627 om.<br> | ||
| + | האחד: V386 האלף<br> | ||
| + | של: O187 שלם<br> | ||
| + | תשעה: O187 om.<br> | ||
| + | ראש האחדים: Lo10785; Mo30 הראש לאחדים<br> | ||
| + | שהוא: O187 om.<br> | ||
| + | שמונים: P1050 השמנים</ref> | ||
|- | |- | ||
| | | | ||
::*If you multiply 9 by 8, the product is 72; 2 is on its left and 7, which corresponds to 70, is on its right. | ::*If you multiply 9 by 8, the product is 72; 2 is on its left and 7, which corresponds to 70, is on its right. | ||
:::<math>\scriptstyle9\times8={\color{blue}{7}}{\color{green}{2}}</math> | :::<math>\scriptstyle9\times8={\color{blue}{7}}{\color{green}{2}}</math> | ||
| − | |style="text-align:right;"|ואם {{#annot:term|185|acHE}}תכפול{{#annotend:acHE}} ט' על ח' יהיה ה{{#annot:term|241|LewN}}מחובר{{#annotend:LewN}}<ref>המחובר: V397 המרובע< | + | |style="text-align:right;"|ואם {{#annot:term|185|acHE}}תכפול{{#annotend:acHE}} ט' על ח' יהיה ה{{#annot:term|241|LewN}}מחובר{{#annotend:LewN}} ע"ב והנה ב' לשמאלו וז' שהוא כנגד ע' לימינו‫<ref>המחובר: V397 המרובע<br> |
| + | ב‫': Lo10785; Mo30; Mu150; P1052; V386 הב‫'<br> | ||
| + | לשמאלו: V171 של שמאלו<br> | ||
| + | שהוא: O187 שהיא; P1052 om.<br> | ||
| + | כנגד: P1029 om.<br> | ||
| + | ע‫': P1052 העין</ref> | ||
|- | |- | ||
| | | | ||
::*If you multiply 9 by 7, the product is 63; 3 is on its left and 6, which corresponds to 60, is on its right. | ::*If you multiply 9 by 7, the product is 63; 3 is on its left and 6, which corresponds to 60, is on its right. | ||
:::<math>\scriptstyle9\times7={\color{blue}{6}}{\color{green}{3}}</math> | :::<math>\scriptstyle9\times7={\color{blue}{6}}{\color{green}{3}}</math> | ||
| − | |style="text-align:right;"|ואם תכפול<ref>תכפול: Lo27153 marg.; W194 om.< | + | |style="text-align:right;"|ואם תכפול ט' על ז' יהיה המחובר ס"ג והנה ג' לשמאלו וו' לימינו שהוא כנגד ס‫'‫<ref>תכפול: Lo27153 marg.; W194 om.<br> |
| + | ט' על: Mu43 שעל<br> | ||
| + | ז‫': Mu43 ו‫'<br> | ||
| + | המחובר: Lo10785; Mo30 המרובע; V171 מרובע<br> | ||
| + | והנה: Lo27153; Mu150; P1052; V386 ויהיה<br> | ||
| + | והנה ג‫': Lo10785; Mo30 וג' הוא<br> | ||
| + | וו‫': Mu43 וז‫'<br> | ||
| + | לימינו שהוא כנגד ס‫': P1050; W152 שהוא כנגד ס' לימינו; B <s>לימינו</s> שהוא כנגד ס‫' <sup>לימינו</sup></ref> | ||
|- | |- | ||
| | | | ||
::*If you multiply 9 by 6, the product is 54, 4 is on its left and 5, which corresponds to 50, is on its right. | ::*If you multiply 9 by 6, the product is 54, 4 is on its left and 5, which corresponds to 50, is on its right. | ||
:::<math>\scriptstyle9\times6={\color{blue}{5}}{\color{green}{4}}</math> | :::<math>\scriptstyle9\times6={\color{blue}{5}}{\color{green}{4}}</math> | ||
| − | |style="text-align:right;"|ואם תכפול ט' על ו' יהיה המחובר<ref>המחובר: P1052 om.< | + | |style="text-align:right;"|ואם תכפול ט' על ו' יהיה המחובר נ"ד והנה ד' לשמאלו וה' שהוא כנגד נ' לימינו‫<ref>המחובר: P1052 om.<br> |
| + | והנה: Lo10785; Mo30; Mu150; P1052; V386 ויהיה<br> | ||
| + | לשמאלו: V171 משמאלו; V397 לשמאל<br> | ||
| + | וה‫': V386 והא‫'<br> | ||
| + | שהוא: V397 om.<br> | ||
| + | נ‫': V386 נון<br> | ||
| + | לימינו: V171 om. V397 מימינו<br> | ||
| + | שהוא ... לימינו: O187 לימינו שהיא חמשים</ref> | ||
|- | |- | ||
| | | | ||
:::Since the number five is mean between the 9 digits, therefore it is called round number, for it circles around itself as its square contains five. | :::Since the number five is mean between the 9 digits, therefore it is called round number, for it circles around itself as its square contains five. | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<div id="five_is_called_round_number"></div>ובעבור כי חשבון חמשה הוא אמצעי בט' מספרים על כן נקרא {{#annot:term|345|1acb}}חשבון עגול{{#annotend:1acb}}<ref group=note>נקרא חשבון עגול: B com נקרא ה' חשבון עגול. ר"ל שימצא במרובעו ואע"פ שבששה ימצא כן אמנם ששה לא ישמור מרובעו במעוקבו כי כשתכפול ו' על ו' יעלו ל"ו ואחר תכפול ו' על ל"ו לא ישאר הל"ו בצורתו. אך ה' פעמ' ה' שהוא כ"ה אם תכפלם על ה' ישמור צורתו עצמו ויעלה קכ"ה וזהו הכפל הגמור כי אחר שכפלנו ארכו על רחבו שהוא השטח נכפלנו בעמקו ונחלק הגבוה לה' חלקי' שוים שכל אחד ה' על ה‫'</ref> כי הוא {{#annot:term|345|ED58}}מתגלגל על עצמו{{#annotend:ED58}}‫<ref group=note>[[פירוש_אנונימי_לספר_המספר#Therefore_five_is_called_round_number.2C_for_it_circles_around_itself|<span style=color:blue>commentary: therefore five is called round number, for it circles around itself</span>]]</ref> כי מרובעו יש בו חמשה‫<ref>חשבון: Mu43 החשבון<br> |
| + | חמשה: V386 הא‫'<br> | ||
| + | מספרים: B; Lo10785; Mo30 המספרים<br> | ||
| + | על: P1051; V397 ועל<br> | ||
| + | על כן: V171 ע"כ<br> | ||
| + | מתגלגל: Lo10785 מ' מתגלגל<br> | ||
| + | מרובעו: O187 om.<br> | ||
| + | כי מרובעו יש בו חמשה: P1050 marg.</ref> | ||
|- | |- | ||
| | | | ||
::*When you multiply 9 by 5, the matter rotates in a circle, for the units are to the right of 9 and the decades to its left; because the product is 45; 5 is on the right side of 9 and the decades are on its left, which is 4 for the 40. | ::*When you multiply 9 by 5, the matter rotates in a circle, for the units are to the right of 9 and the decades to its left; because the product is 45; 5 is on the right side of 9 and the decades are on its left, which is 4 for the 40. | ||
:::<math>\scriptstyle9\times5={\color{green}{4}}{\color{blue}{5}}</math> | :::<math>\scriptstyle9\times5={\color{green}{4}}{\color{blue}{5}}</math> | ||
| − | |style="text-align:right;"|וכאשר תכפול<ref>תכפול: Lo10785 om.< | + | |style="text-align:right;"|וכאשר תכפול ט' על ה' יתגלגל הדבר בעגול כי האחדים יהיו לימין ט' והכללים לשמאלו כי המחובר הוא מ"ה והנה הה' מ{{#annot:term|325|ZJhZ}}פאת{{#annotend:ZJhZ}} ימין ט' והכללים לשמאלו שהוא ד' במקום המ‫'‫<ref>תכפול: Lo10785 om.<br> |
| + | ט' על ה‫': O187 חמשה על ט‫'<br> | ||
| + | בעגול: P1050 top<br> | ||
| + | כי: V171 om.<br> | ||
| + | יהיו: Lo10785; Mo30 om.<br> | ||
| + | ט‫': B; V171 om.<br> | ||
| + | ט' והכללים: Lo10785; Mo30 וט' הכללים<br> | ||
| + | המחובר: Lo10785; Mo30 מחובר ט' על ה‫'<br> | ||
| + | הוא: Lo10785; Mo30 רוא‫'; P1052 יהיו<br> | ||
| + | הה‫': Lo10785; Mo30 ה' הוא; Mu43; Mu150 ה‫'<br> | ||
| + | ט‫': Lo10785; Mo30; Mu150; P1052; V386 הט' הוא האחדים; P1029 ט' הוא האחדים; P1051 ט' והנה האחדים; V171 ט' האחדי‫'; V397 ט' והוא האחדים<br> | ||
| + | הה' מפאת ימין ט‫': O187 מפאת ימין ט' והה' הוא האחדים<br> | ||
| + | והכללים: V397 ו<sup>ה</sup>כללים<br> | ||
| + | הוא ... והכללים: N2627 om.<br> | ||
| + | והכללים לשמאלו שהוא: O187; V171 om.<br> | ||
| + | ד‫': O187; V171 והד‫'; P1029; P1051; V397 הד‫'<br> | ||
| + | המ‫': Lo10785 om.; Mo30; Mu150; P1052; V171; V386 מ‫'</ref> | ||
|- | |- | ||
| | | | ||
::*When you multiply 9 by 4, the product is 36; 3 corresponds to thirty. | ::*When you multiply 9 by 4, the product is 36; 3 corresponds to thirty. | ||
:::<math>\scriptstyle9\times4={\color{green}{3}}{\color{blue}{6}}</math> | :::<math>\scriptstyle9\times4={\color{green}{3}}{\color{blue}{6}}</math> | ||
| − | |style="text-align:right;"|וכאשר תכפול ט' על ד'<ref>ט' על ד': O187 ד' על ט'< | + | |style="text-align:right;"|וכאשר תכפול ט' על ד' יהיה המחובר ל"ו והנה ג' כנגד שלשים‫<ref>ט' על ד‫': O187 ד' על ט‫'<br> |
| + | והנה ג‫': O187 והג‫'<br> | ||
| + | שלשים: V397 הל‫'; P1029 ל' והו' כנגד ששה</ref> | ||
|- | |- | ||
| | | | ||
::*When you multiply 9 by 3, the product is 27; 2 corresponds to twenty. | ::*When you multiply 9 by 3, the product is 27; 2 corresponds to twenty. | ||
:::<math>\scriptstyle9\times3={\color{green}{2}}{\color{blue}{7}}</math> | :::<math>\scriptstyle9\times3={\color{green}{2}}{\color{blue}{7}}</math> | ||
| − | |style="text-align:right;"|וכאשר תכפול ט'<ref>ט': Mu43 om.< | + | |style="text-align:right;"|וכאשר תכפול ט' על ג' יהיה המחובר כ"ז והנה ב' כנגד עשרים‫<ref>ט‫': Mu43 om.<br> |
| + | ט' על ג‫': N2627 ט' לג‫'; O187 ג' על ט‫'<br> | ||
| + | והנה: V386 והוא<br> | ||
| + | ב‫': Lo10785; Mo30; Mu150; P1052; V171; V386 הב‫'<br> | ||
| + | עשרים: Lo10785; Mo30; P1050 העשרים; P1029 כ' וז' כנגד ז‫'; V171 כ"ד</ref> | ||
|- | |- | ||
| | | | ||
::*When you multiply 9 by 2, the product is 18; 1 corresponds to ten. | ::*When you multiply 9 by 2, the product is 18; 1 corresponds to ten. | ||
:::<math>\scriptstyle9\times2={\color{green}{1}}{\color{blue}{8}}</math> | :::<math>\scriptstyle9\times2={\color{green}{1}}{\color{blue}{8}}</math> | ||
| − | |style="text-align:right;"|וכאשר תכפול ט'<ref>ט': Lo10785; Mo30; Mu150 הט'< | + | |style="text-align:right;"|וכאשר תכפול ט' על ב' יהיה המחובר י"ח והנה א' כנגד עשרה‫<ref>ט‫': Lo10785; Mo30; Mu150 הט‫'<br> |
| + | ט' על ב‫': O187 ב' על ט‫'<br> | ||
| + | והנה: Lo10785; Mo30; Mu150; P1052; V386 ויהיה; Mu43 והוא<br> | ||
| + | עשרה: N2627 העשרה; P1029 י' וח' כנגד ח‫'; P1051; V397 עשרה וכאשר תכפול ט' על א' יהיו ט‫'; O187 עשרה וכן נעשה בדומה העשרות צ' על צ' יעלו 00אח וכן כולם וכן נעשה בדומה המאות תת"ק על תת"ק יעלו 0000אח וכך כולם</ref> | ||
|- | |- | ||
|<span style=color:green>Hence the checking scales are based on modulo 9</span> | |<span style=color:green>Hence the checking scales are based on modulo 9</span> | ||
| Line 158: | Line 279: | ||
|- | |- | ||
|Therefore, the scales of a number that is multiplied by itself or by another is 9. | |Therefore, the scales of a number that is multiplied by itself or by another is 9. | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|על כן {{#annot:term|354|DwmZ}}מאזני מספר{{#annotend:DwmZ}} שהוא {{#annot:term|358|oO4Y}}כפול על עצמו{{#annotend:oO4Y}} או על אחר הם ט‫'‫<ref>על: V386 ועל<br> |
| + | על כן: V171 ע"כ<br> | ||
| + | מספר: Lo10785; Mo30; W152 מספר ט‫'; Lo27153 top.; O187; V171 כל מספר; Mu43 המספר<br> | ||
| + | כפול: Mu43; O187 om.<br> | ||
| + | אחר: O187 האחדים; P1029 אחרים<br> | ||
| + | הם: Lo10785; Mo30 הוא; O187 הן<br> | ||
| + | אחר ... ט‫': Mu43 אחד מהם</ref> | ||
|- | |- | ||
| | | | ||
| Line 172: | Line 299: | ||
| | | | ||
:Therefore, the wise men of India have denoted all their numbers by nine and they make forms for the 9 digits. I wrote instead of them: א ב ג ד ה ו ז ח ט [the first nine Hebrew letters] | :Therefore, the wise men of India have denoted all their numbers by nine and they make forms for the 9 digits. I wrote instead of them: א ב ג ד ה ו ז ח ט [the first nine Hebrew letters] | ||
| − | |style="text-align:right;"|‫<ref>P1052 צורה בכתיבת הודו 1 2 3 4 5 6 7 8 9<br>marg. צורת אותיות הודו<br>א ב ג ד ה ו ז ח ט<br> ١ ٢ ٣ ٤ ٥ ٦ ٧ ٨ ٩;<br>V386 צורת כתיבת הודו 1 2 3 4 5 6 7 8 9 10< | + | |style="text-align:right;"|על כן עשו {{#annot:Indian scholars|355|OKOk}}חכמי הודו{{#annotend:OKOk}} כל מספרם על תשעה ועשו {{#annot:term|303|6zsx}}צורות{{#annotend:6zsx}} לט' מספרים<br> |
| − | + | ואני כתבתי במקומם א' ב' ג' ד' ה' ו' ז' ח' ט‫'‫<ref>P1052 צורה בכתיבת הודו 1 2 3 4 5 6 7 8 9<br> | |
| + | marg. צורת אותיות הודו<br>א ב ג ד ה ו ז ח ט<br> | ||
| + | ١ ٢ ٣ ٤ ٥ ٦ ٧ ٨ ٩;<br> | ||
| + | V386 צורת כתיבת הודו 1 2 3 4 5 6 7 8 9 10<br> | ||
| + | על כן: V386 om.<br> | ||
| + | עשו: N2627 עשו <s>זה</s><br> | ||
| + | מספרם: Lo10785; Mo30; Mu150 המספרים<br> | ||
| + | על כן עשו ... על תשעה: O187 om.<br> | ||
| + | מספרים: Lo10785; Mo30 המספרים; Mu43 מספרם; B מספרים והם 1 2 3 4 5 6 7 8 9; N2627 מספרים marg. 1 2 3 4 5 6 7 8 9; O187 מספרים ואלו הם 1 2 3 4 5 6 7 8 9; V171 מספרים אלה ואלה הם ١ ٢ ٣ ٤ ٥ ٦ ٧ ٨ ٩; V397 מספרים marg. 0 9 8 7 6 5 4 3 2 1<br> | ||
| + | תשעה ... מספרים: III ט' אותיות; Lo27153 marg. אילו הם 9 8 7 6 5 4 3 2 1; P1050 ט' אותיות 1 2 3 4 5 6 7 8 9; marg. ועשו צורות לט' מספרי' והם אלו<br> | ||
| + | במקומם: Lo10785; Mo30 תחתיהם; N2627 במקומן; P1029 למקומן<br> | ||
| + | ואני כתבתי במקומם: B ובני ישראל די להם מאותיו' התורה; P1050 marg. כי בני ישראל די להם מאותיו' התורה<br> | ||
| + | א' ... ט‫': P1029 om.; Mu43 א' ב' ג' ד' ה' ו' ז' ח' ט' צורת אנשי הודו<br> | ||
| + | ואני ... ט‫': O187; V171 om.</ref> | ||
|- | |- | ||
| | | | ||
| Line 183: | Line 323: | ||
| | | | ||
:::If you have a number of units and the beginning of the decades, which are tens, always write first the number of the units, then the number of the decades. | :::If you have a number of units and the beginning of the decades, which are tens, always write first the number of the units, then the number of the decades. | ||
| − | |style="text-align:right;"|והנה לעולם אם<ref>אם: V397 om.< | + | |style="text-align:right;"|והנה לעולם אם יש בידך מספר אחדים ותחלת הכללים שהם עשרות יכתוב בתחלה מספר האחדים ואחר כך מספר הכלל‫<ref>אם: V397 om.<br> |
| + | יש בידך: Mu150 marg.; P1050 top; O187 יש בידו<br> | ||
| + | מספר: Lo10785; Mo30 מספרי; P1051 מספר<sup>י</sup>ם; O187; V386 מספרים<br> | ||
| + | יש בידך מספר: III; P1029; V397 מספרם; Mu150; P1052 מספרים; N2627 המספרי‫'; V171 תספרם<br> | ||
| + | אחדים: B; P1050 באחדים<br> | ||
| + | ותחלת: B; V386 לפני; P1051 לפני תחלת; P1050 <sup>נ"א לפני</sup> ותחילת<br> | ||
| + | הכללים: P1029 הכללים ותחלת האחדים<br> | ||
| + | אם יש ... הכללים: B marg. ס"א אם מספרם אחדים ותחילת הכללים<br> | ||
| + | עשרות: B; P1052 העשרות<br> | ||
| + | בתחלה: Lo10785; Mo30; Mu150; P1052 תחלה; V386 תחלת<br> | ||
| + | בתחלה מספר: N2627; P1029 בתחלת המספר<br> | ||
| + | האחדים: Lo10785; Mo30 הכללים Mo30 marg. האחדים; Mu43 אחדים<br> | ||
| + | הכלל: B; P1050; V386 הכללים<br> | ||
| + | P1050 marg. ואם יש בידך אחדי' הרבה ותרצה לכתבם בדרך שלא תטעה שיהיו כללי' כתו' אותם זה למטה מזה וכן בכללי' [..] תרצה לכתוב א' ב' ג' ד' ה' מאחדי' וכן כ' ל' מ' נ' מעשרו' וכן ממאות ואלפי' וכל המדרגות [...]</ref> | ||
|- | |- | ||
| | | | ||
| Line 191: | Line 344: | ||
| | | | ||
:::If there is no number in the units, but it has a number in the second rank, which is the tens, one puts a shape of a wheel first to indicate that there is no number in the first rank and then write the number that he has in the tens afterwards. | :::If there is no number in the units, but it has a number in the second rank, which is the tens, one puts a shape of a wheel first to indicate that there is no number in the first rank and then write the number that he has in the tens afterwards. | ||
| − | |style="text-align:right;"|ואם אין<ref> | + | |style="text-align:right;"|ואם אין לו מספר האחדים ויש לו מספר במעלה השנית שהם {{#annot:term|288|vPba}}העשרות{{#annotend:vPba}} ישים כ{{#annot:term|303|kCK2}}דמות{{#annotend:kCK2}} גלגל<ref group=note>כדמות גלגל: W152 marg. היינו שנקרא בימינו אלה אפס או נול ותמונתו 0</ref> בראשונה להורות כי אין במעלה הראשונה מספר ויכתוב המספר שיש לו בעשרות אחריו‫<ref>ואם אין: Mu43 ואין<br> |
| + | לו: B top; O187 om.<br> | ||
| + | האחדים: B; Lo10785; Mo30; V171 באחדים<br> | ||
| + | מספר האחדים: O187 במספר אחדים<br> | ||
| + | ואחר כך מספר הכלל ואם אין לו מספר האחדים: P1052 om.<br> | ||
| + | מספר: N2627 במספר<br> | ||
| + | שהם: O187 שהיא<br> | ||
| + | העשרות: Lo27153 הע<sup>ש</sup>רות; Lo10785; Mo30; Mu150; P1029; P1051; P1052; V386 עשרות<br> | ||
| + | ישים: P1029 ישים לפניו<br> | ||
| + | גלגל: B גלגל 0; Lo10785; Mo30; Mu150; N2627; P1052 גלגל כזה 0; P1029 גלגל זה 0 הנקרא סיפרא<br> | ||
| + | בראשונה: Mu150; P1029; P1052 om.; V386 בראשונה כזה 0<br> | ||
| + | להורות: P1029 והוא להורות<br> | ||
| + | כי אין: N2627; P1029 שאין<br> | ||
| + | הראשונה: N2627 ראשונה<br> | ||
| + | מספר: Mu150; V171 om.; P1052 מספר <s>במעלה</s><br> | ||
| + | במעלה הראשונה מספר: Lo10785; Mo30 מאומה במעלה הראשנה; V386 מספר במעלה הראשונה<br> | ||
| + | ויכתוב: P1052 ויספור<br> | ||
| + | המספר: Mu43 מספר<br> | ||
| + | בעשרות: Lo10785; Mo30; Mu150; V386 מן העשרות; V397 העשרות<br> | ||
| + | בעשרות אחריו: O187 אחריו בעשרות</ref> | ||
|- | |- | ||
| | | | ||
| Line 200: | Line 372: | ||
| | | | ||
::If his decade consists of the hundreds and the tens, one writes a wheel in the first [rank], then the number of the tens in the second [rank] and the number of hundreds in third [rank]. | ::If his decade consists of the hundreds and the tens, one writes a wheel in the first [rank], then the number of the tens in the second [rank] and the number of hundreds in third [rank]. | ||
| − | |style="text-align:right;"|ואם הכלל שלו<ref>שלו: O187 שיש לו< | + | |style="text-align:right;"|ואם הכלל שלו מהמאות ומהעשרות יכתוב {{#annot:term|205|Ul6o}}גלגל{{#annotend:Ul6o}} בראשונה ואחר כך מספר העשרות בשנית ומספר המאות בשלישית‫<ref>שלו: O187 שיש לו<br> |
| + | מהמאות: B; V171 במאות; Lo10785; Mo30; Mu150; P1052 מן המאות; P1029 <sup>מ</sup>מאות<br> | ||
| + | ומהעשרות: B ובעשרות; Mu43 והעשרות; P1029 ועשרות<br> | ||
| + | מהמאות ומהעשרות: V386 מן העשרות ומן המאות<br> | ||
| + | גלגל: V386 הגלגל<br> | ||
| + | כך: P1052 om.<br> | ||
| + | העשרות: P1052 העשרה<br> | ||
| + | ומספר: Lo10785; Mo30 אח"כ מספר; Mu150 ואחר כך מספר<br> | ||
| + | בשלישית: Mu43 בשלישי</ref> | ||
|- | |- | ||
| | | | ||
| Line 208: | Line 388: | ||
| | | | ||
::If one has a number of thousands [he writes it] in the fourth [rank], the number of tens of thousands in the fifth and the number of the hundreds of thousands in the sixth. | ::If one has a number of thousands [he writes it] in the fourth [rank], the number of tens of thousands in the fifth and the number of the hundreds of thousands in the sixth. | ||
| − | |style="text-align:right;"|ואם<ref>ואם: P1051 וגם marg. נ"ל ואם< | + | |style="text-align:right;"|ואם יש לו מספר אלפים ברביעית ומספר עשרת אלפים בחמישית ומספר מאות אלפים בששית‫<ref>ואם: P1051 וגם marg. נ"ל ואם<br> |
| + | ואם יש לו מספר: Lo10785; Mo30; Mu150; V386 ומספר<br> | ||
| + | ברביעית: N2627; O187 יכתבנו ברביעית<br> | ||
| + | עשרת: N2627 עשרה<br> | ||
| + | מאות: Mo30; Mu43; Mu150; N2627; P1051; P1052; V386 מאת; O187 מאה; P1029; P1050; V171 ק‫'<br> | ||
| + | אלפים: III; Mu150; O187; V386 אלף<br> | ||
| + | בששית: Lo27153 <sup>ב</sup>שישית<br> | ||
| + | ומספר מאות אלפים בששית: Lo10785 om.</ref> | ||
|- | |- | ||
| | | | ||
| Line 216: | Line 403: | ||
| | | | ||
:For 1, 10, 100 repeat as thousands in the fourth, as thousands of thousands in the seventh and as thousands of thousands of thousands in the tenth and so on endlessly. | :For 1, 10, 100 repeat as thousands in the fourth, as thousands of thousands in the seventh and as thousands of thousands of thousands in the tenth and so on endlessly. | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<div id="1_10_100_repeat_as_thousands">כי א'י'ק' יחזור ברביעית לאלפים</div>‫<ref group=note>[[פירוש_אנונימי_לספר_המספר#For_1.2C_10.2C_100_repeat_in_the_thousands|<span style=color:blue>commentary: for 1, 10, 100 repeat in the thousands</span>]]</ref><ref group=note>כי א'י'ק' יחזור ברביעית לאלפים: B com כי אי"ק יחזור באלפים. אי"ק הוא סוג לכל המספרים כי כל מספר הוא אם א' או י' או ק' כי אלף הוא באחד וי' אלפי' ישובו לי' וק' אלפי' ישובו לק'; P1050 marg. וככה נקרא כל אותיות שתמצאם כתו' זו לפני זו כתו' איק בכר גלש כי א' מורה אחדי'. י' מורה עשרו'. ק' מאות. ב' אלפי. כ' עשרת אלפי'. ר'' ק' אלפי'. ג' אלפי אלפי'. ל' י' אלפי' אלפי'. ש' ק' אלפי אלפי' וכן עד עולם. וראה ועשה<br>א י ק ב כ ר ג ל ש<br>9 8 7 6 5 4 3 2 1<br>וכן אם תמצא מספר אחר תקראהו לפי זה הסדר כגון כזה<br>א י ק ב כ<br>1 9 7 5 3<br>וכן אחר כזה<br>א י ק ב כ ר ג ל<br>9 7 0 5 4 0 2 0<br>זהו סדר הקדימה שנלמדת ראשונה</ref> ובשביעית לאלף אלפים ובעשירית לאלף אלפי אלפים וככה עד אין קץ‫<ref>א'י'ק‫': Lo27153; W194 א'י'ק' ב'כ'ר‫'; W152 א'י'ק' ב'כ'ר' ג'ל'ש‫'<br> |
| + | יחזור: Lo27153 חזור marg. ר"ת חוזר<br> | ||
| + | לאלפים: Mu150; V386 אלפים; P1029 om.; P1051 כאלפים<br> | ||
| + | ברביעית לאלפים: B; O187 לאלפים ברביעית; P1050 באלפי' ברביעית; V171 אלפים ב' יחזור <s>בש</s> א'י'ק‫'<br> | ||
| + | כי ... לאלפים: Mo30 marg. כי אי"ק בכ"ר יחזור לאלפים ברביעית ובשביעית<br> | ||
| + | ובשביעית: V171 בשביעית<br> | ||
| + | לאלף: Mo30 אלף<br> | ||
| + | אלפים ובעשירית לאלף: P1050; P1051 om.; V397 <s>[...]</s> ובשמינית לי' אלף אלפים ובתשיעית לאלף אלף אלפי אלפים ובעשרות לאלף<br> | ||
| + | אלפים: Mu150 אלפים ובמעלת י"ג אלף אלפי אלפי אלפים; P1029; V386 אלפים ובמעלת י"ג לאלף אלפי אלפי אלפים<br> | ||
| + | ובעשירית לאלף אלפי אלפים: Mo30; O187; V171 om.<br> | ||
| + | כי א'י'ק' ... אלפי אלפים: Lo10785 om.<br> | ||
| + | וככה: Mu43 om. | ||
| + | קץ: V386 חקר קץ</ref> | ||
|- | |- | ||
| | | | ||
| Line 224: | Line 423: | ||
| | | | ||
:::If there is a number of units and hundreds, but no tens, one writes the number of the units in the first [rank], a wheel in the second [rank] and the number of hundreds in third [rank]. | :::If there is a number of units and hundreds, but no tens, one writes the number of the units in the first [rank], a wheel in the second [rank] and the number of hundreds in third [rank]. | ||
| − | |style="text-align:right;"|ואם יש | + | |style="text-align:right;"|ואם יש לו מספר אחדים ומאות ואין לו עשרות‫<ref group=note>W152 היינו האפס באמצאו למשל 501 וכן הלאה</ref> יכתוב מספר האחדים בראשונה וגלגל בשנית ומספר המאה בשלישית‫<ref>יש: Mu150 <s>אין</s> יש<br> |
| + | עשרות: Lo10785; Mo30; V397 מספר עשרות<br> | ||
| + | מספר אחדים ... יכתוב: P1051 twice<br> | ||
| + | מספר: III; Lo10785; Mo30; P1050 om.; V386 במספר<br> | ||
| + | האחדים: P1051 האחד; V171 אחדים<br>מספר האחדים: V397 <s>מספר המאות</s> מספר האחדים<br> | ||
| + | ומספר המאה: Lo10785; Mo30 ומאה; N2627; O187; P1029; P1050; P1051; P1052; V171; V386; V397 ומספר המאות</ref> | ||
|- | |- | ||
| | | | ||
| Line 232: | Line 436: | ||
| | | | ||
:In this way one puts at first or in the middle two wheels or as much as needed endlessly. | :In this way one puts at first or in the middle two wheels or as much as needed endlessly. | ||
| − | |style="text-align:right;"|ועל זה הדרך<ref>ועל זה הדרך: Lo10785 ועז"ה; V171 וע"ז הדרך; B ועל זה הדרך יעשה לשמור מעלות הגלגל לפי מעלות החשבון שיש לו; N2627 ועל דרך זה< | + | |style="text-align:right;"|ועל זה הדרך ישים שנים גלגלים בראשונה או כפי מה שיצטרך עד אין חקר או באמצע‫<ref>ועל זה הדרך: Lo10785 ועז"ה; V171 וע"ז הדרך; B ועל זה הדרך יעשה לשמור מעלות הגלגל לפי מעלות החשבון שיש לו; N2627 ועל דרך זה<br> |
| + | ישים: O187 יעשה ישים<br> | ||
| + | שנים: P1052 שני<br> | ||
| + | ישים שנים גלגלים בראשונה: B לשום גלגל בראשונה או שני גלגלים; V171 תוכל להשים שנים גלגלים בראשונה<br> | ||
| + | או כפי ... אין חקר: B כפי מה שיצטרך לו בראש<br> | ||
| + | או באמצע: Lo10785; Mo30 om.; Mu150 או ב<sup>א</sup>מצע; O187 וכן באמצע; P1051 בראש או באמצע; B או באמצע וזה דמות הגלגל 0 וטעמו ''כגלגל כקש לפני רוח'' [תהילים פג, י"ד] ואינו אלא לשמור המעלות ובלשון לעז שמו סִיפְרָא<br> | ||
| + | ישים ... או באמצע: P1050 וטעמו ''כגלגל כקש לפני רוח'' ואינו אלא לשמור המעלות ובלע' סיפרא marg. [יע]שה לשמור [מע]לו' הגלגל [לפי] מעלות [ה]גלגל שיש לו [י]שים גלגל [בר]אשונה או [שני] גלגלים [או כפי] מה אז [ש]יצטרך לו [ב]ראשו' או [ב]אמצע וזה דמות גלגל 0<br> | ||
| + | ועל זה הדרך ... או באמצע: B marg. ס"א ועל זה הדרך ישים גלגלים בראשונה או כפי מה שיצטרך עד אין חקר או באמצע</ref> | ||
|- | |- | ||
| | | | ||
| Line 241: | Line 452: | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|ואחר שהזכרתי זה אזכיר<ref>אזכיר: N2627 אזכור< | + | |style="text-align:right;"|ואחר שהזכרתי זה אזכיר שערי זה הספר ונאמר שהם שבעה‫<ref>אזכיר: N2627 אזכור<br> |
| + | זה: Lo10785; Mo30; P1050 om.<br> | ||
| + | הספר: V397 <s>המספר</s> marg. הספר; P1050 המספר<br> | ||
| + | ונאמר: P1029 om.<br> | ||
| + | שהם: Mu150; P1052; V386 כי הם<br>ונאמר שהם: Lo10785; Mo30 והם<br> | ||
| + | שערי זה הספר ונאמר שהם שבעה: O187 שערי זה הספר וזה דמות גלגל 0 וטעמו כגלגל לפני רוח ושערי זה הספר הם שבעה; V171 שערי זה הספר וזה כדמות הגלגל וטעמו כגלגל לפני רוח ושערי זה הספר הם ז‫'</ref> | ||
|- | |- | ||
| | | | ||
*Chapter One: multiplication of a number by itself or by other; multiplication of one number by two numbers or more; multiplication of multiple numbers by multiple [numbers] | *Chapter One: multiplication of a number by itself or by other; multiplication of one number by two numbers or more; multiplication of multiple numbers by multiple [numbers] | ||
| − | |style="text-align:right;"|'''השער הא'''' | + | |style="text-align:right;"|'''השער הא''''‫<ref group=note>הכפל: B marg. מולטיפליקארי</ref> {{#annot:term|156|gpNl}}לכפול חשבון על עצמו{{#annotend:gpNl}}‫<ref group=note>לכפול חשבון על עצמו: B com לכפול חשבון על עצמו. כמו כ"ה על כ"ה</ref> או על אחר‫<ref group=note>או על אחר: B com או על אחר כמו כ"ה על כ"ד</ref> או כפל חשבון אחד על שנים חשבונות‫<ref group=note>או לכפול חשבון אחד על שנים חשבונות: B com או חשבון אחד על שנים. ככפל עשרות על מאות ועשרות</ref> או יותר או כפל חשבונות רבים על רבים‫<ref group=note>או רבים על רבים: B com או רבים על רבים. כמו אחדי' עשרו' ומאות עם אחדי' עשרו' ומאות</ref><ref>הא‫': III; Mu150; N2627; P1050; P1051; V397 הראשון; Lo10785; Mo30 הראשון הוא שער הכפל; B; O187; V171 הא' שער הכפל<br> |
| + | לכפול: P1050 top כפל<br> | ||
| + | על עצמו: O187 בחשבון על עצמו; P1050 על חשבון על עצמו<br> | ||
| + | על: P1051; V397 עם<br> | ||
| + | כפל: B לכפול; Lo27153 <s>כפלי חשבונות</s> <sup>כפלי</sup>; N2627 על כפל; P1051; V397 בהכפל<br> | ||
| + | אחד: P1029 om.<br> | ||
| + | שנים: P1029 שני<br> | ||
| + | חשבונות: Lo10785; Mo30; O187; V171 חשבונים; P1051; V397 om.<br> | ||
| + | יותר: P1051 יתר<br> | ||
| + | כפל: B; P1051 om.<br>או כפל: V397 om.<br> | ||
| + | חשבונות: B; Lo10785; Mo30; V171 om.<br> | ||
| + | על רבים: N2627 om.</ref> | ||
|- | |- | ||
| | | | ||
*Chapter Two: division of decades by units; two decades by units; large decades by small decades; decades and units by units; including a discussion on the checking of multiplication and division | *Chapter Two: division of decades by units; two decades by units; large decades by small decades; decades and units by units; including a discussion on the checking of multiplication and division | ||
| − | |style="text-align:right;"|'''השער הב''''<ref>הב': III; Mo30; Mu150; N2627; P1050; P1051; V397 השני; V171 השני בחלוק; B הב' שער החלוק; O187 הב' בחילוק< | + | |style="text-align:right;"|'''השער הב''''‫<ref group=note>החלוק: B marg. פארטירי</ref> לחלק חשבון כלל על פרט או שנים כללים על פרט אחד או כללים גבוהים על כללים שפלים או כללים ופרטים על פרטים גם אדבר על המאזנים‫<ref group=note>על המאזנים: B com על המאזנים ר"ל האות המעיד המורה על אמתת הכפל או החלוק וחלופו</ref> של שער הכפל והחלוק‫<ref>הב‫': III; Mo30; Mu150; N2627; P1050; P1051; V397 השני; V171 השני בחלוק; B הב' שער החלוק; O187 הב' בחילוק<br> |
| + | לחלק: Lo10785; Mo30 הוא לחלק<br> | ||
| + | כלל: Mu150 כפל<br> | ||
| + | חשבון כלל: P1029 כללי חשבון<br> | ||
| + | פרט: V386 פרט אחד<br> | ||
| + | שנים: III שני<br> | ||
| + | או שנים: P1029; V386 וב‫'; Mu43; Mu150; N2627; P1052; V397 ושנים<br> | ||
| + | על פרט או שנים כללים: P1051 om.<br> | ||
| + | פרט אחד: V397 אחד פרט<br> | ||
| + | כללים: III om.<br> | ||
| + | או: Mu43 om.<br> | ||
| + | ופרטים: P1050 <s>על</s> ופרטים<br> | ||
| + | פרטים: V397 הפרטים<br> | ||
| + | המאזנים: V171 מאזנים<br> | ||
| + | של: N2627; O187; V386 על<br> | ||
| + | שער: O187 שערי<br> | ||
| + | הכפל: V171 והכפל<br> | ||
| + | הכפל והחלוק: Mu150; P1052; V386 החלוק והכפל; P1029 כפל וחלוק</ref> | ||
|- | |- | ||
| | | | ||
*Chapter Three: addition of a number to a number; units to decades or decades to decades | *Chapter Three: addition of a number to a number; units to decades or decades to decades | ||
| − | |style="text-align:right;"|'''השער הג'''' | + | |style="text-align:right;"|'''השער הג''''‫<ref group=note>החבור: B marg. סומארי</ref> בחבור מספר על מספר‫<ref group=note>לחבר מספר אל מספר: B com לחבר מספר על מספר. כגון שתרצה לחבר יחד מספרי' רבי' במספרי' רבי' נכתוב אלו המספרי' בשורות שורות ונחבר האותיו' ביושר כאלו היו אחדים והעולה על עשר אם אין בו אחדים נכתוב גלגל ונשמור א' ואם יש עמו אחדים נכתוב האחדי' ונניח הכלל ונשמרהו. כמו שיתבאר במקומו</ref> פרט עם כלל או כלל עם כלל‫<ref>הג‫': III; Mo30; N2627; P1050; P1051; V171; V397 השלישי; B הג' שער החבור<br> |
| + | בחבור: B; P1029 לחבר<br> | ||
| + | על: Lo10785; Mo30; O187; V397 עם; B; V171 אל<br> | ||
| + | על מספר: P1029 במספר<br> | ||
| + | פרט: V397 כלל עם פרט או פרט<br> | ||
| + | עם: Mu150; P1052; V386 על<br> | ||
| + | עם כלל: P1029 בכלל<br> | ||
| + | עם: Mu150; P1052; V386 על<br> | ||
| + | כלל: Mu43 פרט<br>עם כלל: P1029 בכלל גם אדבר על מאזני שער החבור והמגרעת<br> | ||
| + | פרט עם כלל או כלל עם כלל: O187 כלל עם כלל ופרט עם פרט</ref> | ||
|- | |- | ||
| | | | ||
*Chapter Four: subtraction of a number from a number; units from decades or decades from decades; including a discussion on the checking of addition and subtraction | *Chapter Four: subtraction of a number from a number; units from decades or decades from decades; including a discussion on the checking of addition and subtraction | ||
| − | |style="text-align:right;"|'''השער הד'''' | + | |style="text-align:right;"|'''השער הד''''‫<ref group=note>החסור: B marg. סוטְרַארֵי</ref> {{#annot:term|181|o7zA}}לחסר מספר ממספר{{#annotend:o7zA}}‫<ref group=note>לחסר מספר ממספר: B com לחסר מספר ממספר. כלו' לגרוע מספר ממספר אחר רב ממנו ולדעת הנשאר</ref> פרט מכלל או כלל מכלל גם אדבר על מאזני שער החבור והמגרעת‫<ref>הד‫': III; Mo30; Mu150; N2627; P1050; P1051; V171; V397 הרביעי; B הד' שער החסור<br> |
| + | לחסר: Mu43 להסר; P1050 בחסר; V171 לחסור<br> | ||
| + | מספר: Lo10785 twice; O187 <s>מ</s>מספר<br> | ||
| + | פרט: Mu150; V386 פרט או<br> | ||
| + | מכלל: Mu43 om.<br> | ||
| + | אדבר: Mu150 om.<br> | ||
| + | שער: O187; P1051; V171; V397 שערי<br> | ||
| + | מאזני שער: Mu150 שער מאזני<br> | ||
| + | החבור: Lo10785; Mo30 החלוק<br> | ||
| + | גם אדבר ... והמגרעת: P1029 om.</ref> | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|'''השער הה''''<ref>הה': III; Mo30; N2627; O187; P1051; V397 החמישי; Mu150 החמשה< | + | |style="text-align:right;"|'''השער הה'''' על השברים והם על דרכים רבים שלמים על שלמים ונשברים עמהם או שלמים ונשברים עם שלמים ונשברים למיניהם או שברים עם שברים או שברים על שברי שברים או שברי שברים על שברי שברים בין לכפול בין לחלק בין לחבר בין לגרוע ומאזניהם‫<ref>הה‫': III; Mo30; N2627; O187; P1051; V397 החמישי; Mu150 החמשה<br> |
| + | על השברים: Lo10785; Mo30 ידבר על השברים; B שער השברים<br> | ||
| + | על: Mu43 om.<br> | ||
| + | על דרכים: Lo10785; Mo30 מינים<br> | ||
| + | על: B עם marg. נ' על; Lo10785; Mo30; Mu150; O187; P1029; P1051; P1052; V171; V386; V397 עם<br> | ||
| + | שלמים: P1050 שלמים או שלמים<br> | ||
| + | ונשברים: P1050; P1051; V397 ושברים<br> | ||
| + | ונשברים: P1051; V397 ושברים; V171 om.<br> | ||
| + | עם: B עם marg. נ' על; N2627 על<br> | ||
| + | שלמים: V171 ונשלמים<br> | ||
| + | ונשברים: Mu43; N2627; W152; W194 ונשבריהם<br> | ||
| + | ונשברים עמהם או שלמים ונשברים עם שלמים ונשברים: Lo10785; Mo30 ושברים עם שלמים ושברים<br> | ||
| + | למיניהם: Mu150; P1052; V386 הם למיניהם; V397 עמהם למיניהם<br> | ||
| + | עם: B; N2627 על<br> | ||
| + | או שברים: Mu43 om.<br> | ||
| + | על: Lo10785; Mo30; P1051; P1052 עם<br> | ||
| + | שברי: P1051 שברים<br> | ||
| + | או שברים על שברי שברים: V397 marg<br> | ||
| + | שברים: Mu150 marg.<br>שברי שברים: P1052 שברי<s>ם</s> שברים<br> | ||
| + | על: Lo10785; Mo30; P1052; V397 עם<br> | ||
| + | שברי: P1050 שברי <s>שברי</s>; P1052 om.<br> | ||
| + | או שברי שברים על שברי שברים: Lo27153; P1051 om.<br> | ||
| + | בין לחבר: P1051; V397 om.<br> | ||
| + | בין לחבר בין לגרוע: Lo10785; Mo30 בין לגרוע בין לחבר<br> | ||
| + | ומאזניהם: P1052 om.</ref> | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|'''השער הו''''<ref>הו': III; Mo30; Mu150; N2627; P1050; P1051; V397 השישי< | + | |style="text-align:right;"|'''השער הו'''' בערכים והוא שער נכבד מאד כי ממנו יוכל להוציא רובי השאלות הקשות ורוב הראיות מחכמת המזלות יצאו מזה הערך‫<ref>הו‫': III; Mo30; Mu150; N2627; P1050; P1051; V397 השישי<br> |
| + | בערכים: B שער הערכים; Lo10785; Mo30; Mu43; O187; P1029; P1051; V171; V397 בערכין<br> | ||
| + | מאד: Lo10785; Mo30 om.; V386 ממנו<br> | ||
| + | יוכל: Lo10785; Mu43; Mu150; O187; P1029; P1052; V386 נוכל; B יוכל האדם<br> | ||
| + | להוציא: III להוציא האדם<br> | ||
| + | רובי: Lo10785; Mo30; O187 רוב; V386 כל; Mu150 om.<br> | ||
| + | ורוב: P1029 והוא marg. ורוב<br> | ||
| + | ורוב הראיות: B ורוב <sup>ה</sup>ראיות; Lo10785; Mo30 והראיות<br> | ||
| + | מחכמת: Mu43 מחכמות<br> | ||
| + | מזה: B מן marg. נ' מזה; P1051 ממנו<br> | ||
| + | הערך: Mu43 השער<br> | ||
| + | מזה הערך: Lo10785; Mo30; V171 מהערך</ref> | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|'''השער הז''''<ref>הז': III; Mo30; Mu150; N2627; P1050; P1051; V397 השביעי<br>השער הז': V171 שער הז'< | + | |style="text-align:right;"|'''השער הז'''' על שרשי המרובעים והמאזנים שלהם כי הם רבים וחכמת המדות תלויה בשער הזה וזה השער חמור מכל השערים ואין כח במשכיל לדעת קדרות המאורות אם לא ילמד זה השער ויתרי קשתי העגול יצאו מהשער הזה‫<ref>הז‫': III; Mo30; Mu150; N2627; P1050; P1051; V397 השביעי<br> |
| + | השער הז‫': V171 שער הז‫'<br> | ||
| + | על שרשי: Mu43 והוא שער נכבד מאד כי ממנו נוכל להוציא על שרשי; B שער השרשי‫'<br> | ||
| + | והמאזנים: B וכל המאזנים<br> | ||
| + | רבים: P1029 top<br> | ||
| + | הם רבים: B רבים הם<br> | ||
| + | תלויה: P1029; P1052 תלוי<br> | ||
| + | בשער הזה: Lo10785; Mo30; Mu43; P1050 בזה השער; Mu150; P1029; P1052 בשער זה<br> | ||
| + | וזה: V171 om.<br> | ||
| + | וזה השער: P1051; V397 וזה שער; B והוא<br> | ||
| + | חמור: Mu150 האמור<br> | ||
| + | השערים: Lo10785 השערים אשר; Mo30 השערים אשר לפניו<br> | ||
| + | ואין: Lo10785 om.<br> | ||
| + | במשכיל: P1051 להשכיל; P1052 למשכיל<br> | ||
| + | כח במשכיל: Lo10785; Mo30 בכח משכיל<br> | ||
| + | קדרות: P1052 סדרי קדרות; O187 קדר<sup>ו</sup>ת<br> | ||
| + | ילמד: Lo10785; N2627; O187; P1029; P1051; P1052; V171; V386 ילמוד<br> | ||
| + | זה: Lo10785; Mo30 תחלה זה<br> | ||
| + | זה השער: P1029 שער זה; V171 השער הזה<br> | ||
| + | ויתרי: V386 והיתרים; Mu43 ויתר ידוע<br> | ||
| + | קשתי: O187 קשתות; V171 קשת; V386 וקשתי<br> | ||
| + | העגול: Mu43; Mu150; P1029; P1051 זה העגול<br> | ||
| + | יצאו: O187; P1052 יצא<br> | ||
| + | מהשער הזה: III; N2627; P1050 מזה השער; P1029; P1052 משער זה<br> | ||
| + | יצאו מהשער הזה: V171 om.</ref> | ||
|- | |- | ||
| | | | ||
| Line 285: | Line 606: | ||
| | | | ||
:I have already mentioned what the ranks of the number are. When you have two numbers to multiply a decade by a decade, be it by itself, or by another, look for their analogous in the first rank, see how much is the product of the multiplication of one by the other and keep it. Then, see how many ranks the two numbers are, whether they are in the same rank or two [different] ranks, and know what the sum of the numbers of the two ranks is. Always subtract one for the foundation and put the reserved number in the remaining number of the ranks. | :I have already mentioned what the ranks of the number are. When you have two numbers to multiply a decade by a decade, be it by itself, or by another, look for their analogous in the first rank, see how much is the product of the multiplication of one by the other and keep it. Then, see how many ranks the two numbers are, whether they are in the same rank or two [different] ranks, and know what the sum of the numbers of the two ranks is. Always subtract one for the foundation and put the reserved number in the remaining number of the ranks. | ||
| − | |style="text-align:right;"|כבר הזכרתי איך הם<ref group=I>הם: O187 הן< | + | |style="text-align:right;"|כבר הזכרתי איך הם מעלות המספר והנה כשיבואו לך שנים מספרים לכפול כלל על כלל<br> |
| − | + | בין שיהיה על עצמו<br> | |
| − | + | או על אחר<br> | |
| − | + | בקש דמיונו במעלה הראשונה וראה כמה המחובר מכפל זה על זה ושמור אותו<br> | |
| − | + | ואחר כך ראה כמה מעלות שני החשבונות בין שיהיה במעלה אחת או בשתים מעלות ודע כמה המחובר ממספר שתים מעלות וגרע לעולם אחד למוסד<br> | |
| − | + | ובקש במספר המעלה הנשאר במספר השמור‫<ref group=I>הם: O187 הן<br> | |
| + | המספר: P1051 <s>המעלות</s> המספר; P1050; W194 מספר<br> | ||
| + | והנה: P1050; III הנה; P1051 וזה<br> | ||
| + | כשיבואו: P1051 כשיבהו marg. נ"ל כשיהיו; P1052; W152 כשיבא<br> | ||
| + | שנים: Lo27153; Mu150; O187; P1052 שני<br> | ||
| + | לכפול: P1052 <s>לכלו</s> לכפ<sup>ו</sup>ל<br> | ||
| + | על: V397 עם<br> | ||
| + | שיהיה: Mu150; P1052 om.<br> | ||
| + | על עצמו: P1050; III על עצמו כמו כ' על כ‫'<br> | ||
| + | או: P1052 בין<br> | ||
| + | על אחר: P1050; III על אחר כמו ‫(Lo כגון)‫ כ' על ל‫';<br> | ||
| + | V397 על אחר כמו כ' על ב' או כ' על ל‫'<br> | ||
| + | בקש: P1051 בקש<s>נו</s><br> | ||
| + | V398 begin | ||
| + | כמה: P1050 om.<br> | ||
| + | מכפל: P1052 ותכפול<br> | ||
| + | ושמור: P1052 ותשמור<br> | ||
| + | אותו: V398 om.<br> | ||
| + | ואחר כך: Mo30 אח"כ<br> | ||
| + | שני: P1052 שתי<br> | ||
| + | שני החשבונות: Mo30; O187; P1051; V397 שנים החשבונים<br> | ||
| + | שיהיה: Mo30; O187; P1029 שיהיו; Mu150; V397 שהיה<br> | ||
| + | או: Mu150 בין<br> | ||
| + | בשתים: Mo30 בשני; Mu150; P1052 בשתי; P1051; V397 בשנים<br> | ||
| + | ממספר: P1051 ממספרם<br> | ||
| + | שתים: O187 השתים<br> | ||
| + | ממספר שתים: III משתים; Lo27153 <s>משנים</s> <sup>משתים</sup>; P1050 <sup>במספר</sup> משתים<br> | ||
| + | מעלות: P1050 <sup>ה</sup>מעלות<br> | ||
| + | שתים מעלות: Mo30; P1051; P1052; V397 שתי המעלות; Mu150 שני המעלות<br> | ||
| + | אחד: P1050 אחת<br> | ||
| + | במספר: Mo30; O187 המספר<br> | ||
| + | המעלה: P1050 מעלת<br> | ||
| + | במספר המעלה: III מספר מעלות; O187 הנשאר המעלה<br> | ||
| + | הנשאר: Mu150 הנ<sup>א</sup>שר הנשאר; O187; V397 הנשארת<br> | ||
| + | במספר: V397 מהמספר; III om.<br> | ||
| + | השמור: III וכפי מספר המעלות כן תכתוב השמור (W152 השמור תכתוב)</ref> | ||
|- | |- | ||
|I will discuss the meaning of the foundation in my discussion about the secret of the one, with the help of God. | |I will discuss the meaning of the foundation in my discussion about the secret of the one, with the help of God. | ||
| − | |style="text-align:right;"|ועוד אדבר על טעם<ref group=I>על טעם: P1052 בטעמי< | + | |style="text-align:right;"|ועוד אדבר על טעם המוסד בדברי על סוד האחד בע"ה‫<ref group=I>על טעם: P1052 בטעמי<br> |
| + | המוסד: V398 <s>אחר</s> המוסד<br> | ||
| + | האחד: P1029 אחת האחד; P1050 <sup>ה</sup>אחד<br> | ||
| + | ועוד ... האחד: Mo30 marg.<br> | ||
| + | בע"ה: Mo30; O187 om.</ref> | ||
|- | |- | ||
| | | | ||
:*{{#annot:30×200|156|8PCw}}Example: we wish to multiply thirty by two hundred. | :*{{#annot:30×200|156|8PCw}}Example: we wish to multiply thirty by two hundred. | ||
::<math>\scriptstyle30\times200</math> | ::<math>\scriptstyle30\times200</math> | ||
| − | |style="text-align:right;"|<big>דמיון</big> רצינו<ref group=I>רצינו: P1029 מצינו</ref> | + | |style="text-align:right;"|<big>דמיון</big> רצינו לכפול שלשים על מאתים{{#annotend:8PCw}}‫<ref group=I>רצינו: P1029 מצינו</ref> |
|- | |- | ||
| | | | ||
::The number that is analogous to thirty is three and the number that is analogous to two hundred is two. We multiply 2 by 3, the result is six and this is the reserved number. We seek the ranks: thirty is of the second rank, which are the tens; we take two for it. Since the two hundred is of the third rank, for they are the hundreds, we take three for it and add the two to them; they are five. We subtract one to the foundation; four remain. As we already know that the fourth rank is for the thousand and the reserved number is six, the result is six thousand. | ::The number that is analogous to thirty is three and the number that is analogous to two hundred is two. We multiply 2 by 3, the result is six and this is the reserved number. We seek the ranks: thirty is of the second rank, which are the tens; we take two for it. Since the two hundred is of the third rank, for they are the hundreds, we take three for it and add the two to them; they are five. We subtract one to the foundation; four remain. As we already know that the fourth rank is for the thousand and the reserved number is six, the result is six thousand. | ||
| − | |style="text-align:right;"|והנה<ref group=I>והנה: Mo30; Mu150; P1052 הנה< | + | |style="text-align:right;"|והנה דמיון שלשים שלשה ודמיון מאתים שנים<br> |
| − | כפלנו ב | + | כפלנו ב' על ג' והנה עלו ששה וזהו החשבון השמור<br> |
| − | + | ונשוב לבקש המעלות והנה שלשים מהמעלה השנית שהם עשרות והנה נקח לו שנים<br> | |
| − | + | ובעבור כי המאתים מהמעלה השלישית כי הם מאות נקח לו שלשה ונחבר עליו השנים ויהיה חמשה<br> | |
| − | + | נחסר אחד למוסד ישארו ארבעה וכבר ידענו כי המעלה הרביעית היא לאלף<br> | |
| − | + | והמספר השמור היה ששה והנה העולה ששת אלפים‫<ref group=I>והנה: Mo30; Mu150; P1052 הנה<br> | |
| + | שלשים: V397 השלשים<br> | ||
| + | שלשים שלשה: P1052 שלשים הוא שלשה; W152 ל‫' <sup>הוא</sup> ג‫'<br> | ||
| + | ודמיון: V397; V398; W152; W194 דמיון<br> | ||
| + | מאתים שנים: P1052 מאתים הוא שנים; W152 ר‫' <sup>הוא</sup> ב‫'<br> | ||
| + | כפלנו ב‫': V398 marg.<br> | ||
| + | והנה: Mo30; P1052 om.<br> | ||
| + | עלו: Mu150; P1052 יעלו<br> | ||
| + | וזהו: III; Mu150 וזה<br> | ||
| + | החשבון השמור: P1052 חשבון שמור<br> | ||
| + | מהמעלה: Mu150; P1029; P1052 ממעלה<br> | ||
| + | עשרות: P1050; III העשרות<br> | ||
| + | לו: P1052 אותו<br> | ||
| + | שנים: Mu150 ב' מעלות<br> | ||
| + | המאתים: Mo30 מאתים<br> | ||
| + | כי המאתים: P1050 שהמאתים<br> | ||
| + | מהמעלה: P1052 ממעלה<br> | ||
| + | כי הם: P1029 שהם<br> | ||
| + | שלשה: Mu150 שלשה מעלות<br> | ||
| + | עליו: Mo30; O187; P1029; P1050 אליו<br> | ||
| + | השנים: P1052 ב‫'<br> | ||
| + | ויהיה: Mo30; Mu150; P1050; P1051; P1052; III ויהיו<br> | ||
| + | נחסר: Mu150 ונחסר<br> | ||
| + | אחד: Lo27153 om.<br> | ||
| + | ישארו: Mo30; P1051 וישארו; O187 ישאר; V398 נשארו<br> | ||
| + | ידענו: Mo30 אמרנו; Mu150 הזכרנו; O187 ידעת<br> | ||
| + | הרביעית: O187 <sup>ה</sup>רביעית<br> | ||
| + | לאלף: Mu150; P1052 לאלפים<br> | ||
| + | השמור: O187 om.; P1051 השמורי<sup>ם</sup><br> | ||
| + | היה: Mo30 הוא; P1029; P1051; V397 הם; P1050; III om.<br> | ||
| + | ששה: Lo27153 <s>ה</s>ששה<br> | ||
| + | והנה: Mo30 ויהיה<br> | ||
| + | והנה העולה: P1051 והעולה<br> | ||
| + | ששת אלפים: P1029 הם ו' אלפים כזה 000ו; Mu150 ו' אלפים כזו הצורה; P1052 ו' אלפים כמו הצורה</ref> | ||
|- | |- | ||
| | | | ||
:*{{#annot:200×700|156|gpzH}}Another example: we wish to multiply two hundred by seven hundred. | :*{{#annot:200×700|156|gpzH}}Another example: we wish to multiply two hundred by seven hundred. | ||
::<math>\scriptstyle200\times700</math> | ::<math>\scriptstyle200\times700</math> | ||
| − | |style="text-align:right;"|<big>דמיון<ref group=I>דמיון: P1029 ודמיו'< | + | |style="text-align:right;"|<big>דמיון אחר</big> בקשנו לכפול מאתים על שבע מאות{{#annotend:gpzH}}‫<ref group=I>דמיון: P1029 ודמיו‫'<br> |
| + | אחר: P1050 om.<br> | ||
| + | בקשנו: P1050 רצינו</ref> | ||
|- | |- | ||
| | | | ||
::We multiply two by seven; it is 14 and this is the reserved. two hundred is of the third rank and 7 hundred is also of to the third rank; we take for them 6 and subtract one, it is 5. The beginning of the fifth rank is ten thousand and the reserved number is 14, so we take ten thousand of this number, the result is one hundred thousand and forty thousand. | ::We multiply two by seven; it is 14 and this is the reserved. two hundred is of the third rank and 7 hundred is also of to the third rank; we take for them 6 and subtract one, it is 5. The beginning of the fifth rank is ten thousand and the reserved number is 14, so we take ten thousand of this number, the result is one hundred thousand and forty thousand. | ||
| − | |style="text-align:right;"|והנה כפלנו שנים על שבעה<ref group=I>והנה ... ז': Mo30 marg.< | + | |style="text-align:right;"|והנה כפלנו שנים על שבעה והנה י"ד והוא השמור<br> |
| − | + | והנה מאתים מהמעלה השלישית וז' מאות גם כן מהמעלה השלישית נקח להם ששה ונחסר אחד הנה חמשה<br> | |
| − | + | וראש המעלה החמישית עשרת אלפים והשמור היה י"ד<br> | |
| − | + | הנה נקח במספר הזה עשרת אלפים ויהיה העולה מאה אלף וארבעים אלף‫<ref group=I>והנה ... ז‫': Mo30 marg.<br> | |
| + | והנה: Mo30 עלו; mu150 והוא; O187 הם; P1029; P1051; V397 הנה; P1050; P1052; W152 והם<br> | ||
| + | והוא: O187 הוא; P1050 והנה<br> | ||
| + | מאתים: Mu150; P1052 המאתים; W152 הר‫'<br> | ||
| + | גם כן: Mo30; O187 om.; P1051 אם כן<br> | ||
| + | וז' מאות גם כן: P1052 וכן ז' מאות<br> | ||
| + | מהמעלה השלישית: Mu150; P1051; V398 מהשלישית<br> | ||
| + | נקח: Mo30 והנה נקח<br> | ||
| + | להם: O187; V397 לו<br> | ||
| + | נקח להם: P1051 נקרא לו; W152 ויהיו<br> | ||
| + | ונחסר: Mu150 ונחסר להם; W152 נחסר<br> | ||
| + | אחד: O187 ממנו אחד; V397 אחת; Mu150; P1052; W152 אחד למוסד<br> | ||
| + | הנה: Mo30; Mu150; P1052 והנה; W152 ישארו; Lo27153; W194 הרי<br> | ||
| + | וראש: P1051 נראה; V397 נראה ראש<br> | ||
| + | המעלה: O187 מעלה<br> | ||
| + | וראש המעלה: P1050; III והמעלה<br> | ||
| + | החמישית: Mu150 החמשית מעלת הרבבות שהם; P1052 החמישית מעלת הרבואות שהם; V397 חמישית<br> | ||
| + | עשרת: Mo30 הוא עשרת; P1052 עשר<sup>ו</sup>ת; V397 הנה עשרת<br> | ||
| + | היה: Mo30 הוא<br> | ||
| + | במספר: P1052 למספר<br> | ||
| + | הזה: P1029 זה<br> | ||
| + | עשרת: P1052 עשרות<br> | ||
| + | עשרת אלפים: V398 ויהיה העולה עשרת אלפים<br> | ||
| + | הנה ... אלפים: Mo30 om.<br> | ||
| + | והשמור היה ... עשרת אלפים: P1050; III om.<br> | ||
| + | ויהיה: Mo30 א"כ יהיה; W152 ויהיו<br> | ||
| + | העולה: P1050; III om.<br> | ||
| + | מאה: Mu150; P1051; P1052; V397; III מאת<br> | ||
| + | אלף: P1029; P1051; V397 אלפים; P1050 om.<br> | ||
| + | אלף: P1050 אלף ר"ל ק"מ אלפי‫'<br> | ||
| + | מאה אלף וארבעים אלף: O187 ק"מ אלפים<br> | ||
| + | Mu150 כזו הצורה; P1052 כזאת הצורה</ref> | ||
|- | |- | ||
| | | | ||
:You can proceed endlessly according to this procedure. | :You can proceed endlessly according to this procedure. | ||
| − | |style="text-align:right;"|ועל<ref group=I>ועל: Mu150; P1029; P1052 והנה על< | + | |style="text-align:right;"|ועל זה הסדר תוכל לעשות עד אין קץ‫<ref group=I>ועל: Mu150; P1029; P1052 והנה על<br> |
| + | הסדר: Lo27153 <s>הדרך</s> הסדר<br> | ||
| + | קץ: O187 מספר</ref> | ||
|- | |- | ||
| | | | ||
| Line 331: | Line 759: | ||
| | | | ||
:If there are two numbers whose distance from a decade is as much as the distance of two other numbers, only that the one is deficient and the other exceeding, know how much the square of the decade, always subtract from it the square of the excess and the deficit and the remainder is the sought number. | :If there are two numbers whose distance from a decade is as much as the distance of two other numbers, only that the one is deficient and the other exceeding, know how much the square of the decade, always subtract from it the square of the excess and the deficit and the remainder is the sought number. | ||
| − | |style="text-align:right;"|ואם היו שנים<ref group=I>שנים: P1050 שני; P1052 השנים< | + | |style="text-align:right;"|ואם היו שנים מספרים מרחקם מחשבון כלל כמרחק שנים מספרים אחרים רק האחד במגרעת והשני בתוספת<br> |
| − | + | דע כמה מרובע מספר הכלל וגרע ממנו לעולם מרובע החשבון היתר והחסר והנשאר הוא החשבון המבוקש‫<ref group=I>שנים: P1050 שני; P1052 השנים<br> | |
| + | מרחקם: Mo30 אשר מרחקם; Mu150 מחזיקים<br> | ||
| + | מחשבון: O187 מן חשבון<br> | ||
| + | שנים: P1029; P1052 שני<br> | ||
| + | שנים מספרים: O187 שוה מהמספרים<br> | ||
| + | אחרים: Mo30 om.<br> | ||
| + | רק: Mo30 אך<br> | ||
| + | האחד במגרעת והשני בתוספת: Mo30 האחד בתוספת והשני במגרעת<br> | ||
| + | כמה: O187 כמו<br> | ||
| + | מרובע: Mo30; P1029; P1050; V398; W152; W194 om.<br> | ||
| + | מרובע מספר: O187 מספר מרובע; P1052 מספר <sup>מרובע</sup><br> | ||
| + | הכלל: Mo30 הכלל המבוקש; P1052 הכולל<br> | ||
| + | החשבון היתר והחסר: P1051; V397 חשבון החסר והיתר; O187 חשבון היתר והחסר<br> | ||
| + | והנשאר הוא: W152 והוא<br> | ||
| + | החשבון: Mo30; III; P1051; P1052 om.; Mu150; O187 חשבון</ref> | ||
|- | |- | ||
| | | | ||
| − | :*{{#annot:29×31|156|jFgw}}Example: we wish to multiply 29 by 31. | + | :*<div id="29_by_31"></div>{{#annot:29×31|156|jFgw}}Example: we wish to multiply 29 by 31. |
::<math>\scriptstyle29\times31</math> | ::<math>\scriptstyle29\times31</math> | ||
| − | |style="text-align:right;"|<big>דמיון</big> רצינו לכפול כ"ט על ל"א‫<ref group=I>ל"א: P1029 <s>לא</s> ל"א</ref> | + | |style="text-align:right;"|<big>דמיון</big> רצינו לכפול כ"ט על ל"א{{#annotend:jFgw}}‫<ref group=note>[[Excerpts_Attributed_to_Immanuel_Bonfils#Example:_we_wish_to_multiply_29_by_31|<span style=color:blue>commentary: example: we wish to multiply 29 by 31</span>]]</ref> |
| + | <ref group=I>ל"א: P1029 <s>לא</s> ל"א</ref> | ||
|- | |- | ||
| | | | ||
::The decade is thirty, its square is 9 hundred, because three by three are nine; the excess and the deficit is one, its square one; we subtract it from the square of the decade, the remainder is the sought after, which is 899. | ::The decade is thirty, its square is 9 hundred, because three by three are nine; the excess and the deficit is one, its square one; we subtract it from the square of the decade, the remainder is the sought after, which is 899. | ||
| − | |style="text-align:right;"|והנה חשבון<ref group=I>חשבון: Mo30; O187; P1050; P1051; V397 החשבון< | + | |style="text-align:right;"|והנה חשבון הכלל הוא שלשים ומרובעו ט' מאות כי שלשה על שלשה הם תשעה ‫<ref group=I>MS N2624 begin.</ref>והיתרון והחסרון הוא אחד ומרובעו אחד חסרנום ממרובע הכלל והנשאר הוא המבוקש והוא תתצ"ט‫<ref group=I>חשבון: Mo30; O187; P1050; P1051; V397 החשבון<br> |
| + | הוא: Mo30; P1052 om.<br> | ||
| + | ומרובעו: Mu150 ומרובע ל' פי' ל' פעמים ל' הם; P1052 ומרובע ל' פעמים ל' הם<br> | ||
| + | שלשה: P1051 א‫'<br> | ||
| + | הם: Mo30; P1029; P1051; V397; III om.<br> | ||
| + | והחסרון: P1050 twice; W152 om.<br> | ||
| + | והיתרון והחסרון: Lo27153; Mu150; P1029; P1052; W194 והחסרון והיתרון<br> | ||
| + | הוא: P1029 om.<br> | ||
| + | אחד: V397 הא‫'<br> | ||
| + | אחד: Mu150 <s>אחד</s> א‫'; P1051 אחד <s>ממר</s><br> | ||
| + | חסרנום: Mo30 נחסרנו; Mu150 חסר א‫'; O187 וחסרנו א‫'; P1029 וסרנוהו; P1050 <sup>נ</sup>חסרנו<s>הו</s>; P1051 וחסרנום; P1052 חסרנו; V397 נחסרנום; W152 חסרנוהו<br> | ||
| + | ממרובע: Mo30 מחשבון<br> | ||
| + | הכלל: Mo30 כלל<br> | ||
| + | והוא: P1029 שהוא; P1052 והנה; V398; III; Mu150 om.<br> | ||
| + | תתצ"ט: Mu150 כזה טטח; V398; III om. (Lo27153 add. marg.)<br> | ||
| + | והוא תתצ"ט: P1050 marg. זהו תתצ"ט</ref> | ||
|- | |- | ||
| colspan=2| | | colspan=2| | ||
| Line 349: | Line 807: | ||
:*{{#annot:66×54|156|mnFQ}}Another example: we wish to multiply 66 by 54. | :*{{#annot:66×54|156|mnFQ}}Another example: we wish to multiply 66 by 54. | ||
::<math>\scriptstyle66\times54</math> | ::<math>\scriptstyle66\times54</math> | ||
| − | |style="text-align:right;"|<big>דמיון אחר</big> רצינו לכפול ס"ו על נ"ד‫<ref group=I>נ"ד: P1029 <s>נ"ט</s> <sup>נ"ד</sup><br>ס"ו על נ"ד: Mu150 נ"ד על ס"ו</ref> | + | |style="text-align:right;"|<big>דמיון אחר</big> רצינו לכפול ס"ו על נ"ד{{#annotend:mnFQ}}‫<ref group=I>נ"ד: P1029 <s>נ"ט</s> <sup>נ"ד</sup><br>ס"ו על נ"ד: Mu150 נ"ד על ס"ו</ref> |
|- | |- | ||
| | | | ||
::The decade is 60, the deficit and the excess is 6, the square of the decade is 3 thousand and 600. We subtract from it 36, which is the square of the deficit and the excess, the remainder is the sought after. | ::The decade is 60, the deficit and the excess is 6, the square of the decade is 3 thousand and 600. We subtract from it 36, which is the square of the deficit and the excess, the remainder is the sought after. | ||
| − | |style="text-align:right;"|והנה חשבון<ref group=I>חשבון: Lo27153 <s>ה</s>חשבון; V397 החשבון< | + | |style="text-align:right;"|והנה חשבון הכלל ס' והחסרון והיתרון הוא ששה והנה מרובע הכלל ג' אלפים ות"ר נחסר ממנו ל"ו שהוא מרובע החסרון והיתרון והנשאר הוא המבוקש‫<ref group=I>חשבון: Lo27153 <s>ה</s>חשבון; V397 החשבון<br> |
| + | ס‫': Mo30 הוא ס‫'<br> | ||
| + | והחסרון והיתרון: Mo30 והיתרון והחסרון<br> | ||
| + | הוא: V398 הנה<br> | ||
| + | הכלל: P1029 הכלל ס' על ס‫'; V397 הכולל; W152; W194 הכל<br> | ||
| + | מרובע: P1051; V397 om.<br> | ||
| + | החסרון והיתרון: V397 היתרון והחסרון; P1029 החסרון והיתרון שהוא ו' על ו‫'<br> | ||
| + | ל"ו שהוא מרובע החסרון והיתרון: Mo30 מרובע החסרון והיתרון והוא ל"ו<br> | ||
| + | והנשאר: Mu150 והנש<sup>א</sup>ר; V397 והשאר<br> | ||
| + | המבוקש: O187 המבוקש ג' אלפים ותקס"ד; P1029 המבוקש שהוא ג' אלפים ותקס"ד</ref> | ||
|- | |- | ||
| colspan=2| | | colspan=2| | ||
| Line 361: | Line 828: | ||
:*{{#annot:250×350|156|jWUi}}Another example: one number is 250 and the other number 350. | :*{{#annot:250×350|156|jWUi}}Another example: one number is 250 and the other number 350. | ||
::<math>\scriptstyle250\times350</math> | ::<math>\scriptstyle250\times350</math> | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<big>דמיון אחר</big> {{#annot:term|35|PgHE}}המספר{{#annotend:PgHE}} האחד ר"נ והמספר האחר ש"נ{{#annotend:jWUi}}‫<ref group=I>III example is missing (Lo27153 added marg.)<br> |
| + | דמיון אחר: P1050 ד"א<br> | ||
| + | האחר: Mu150; O187; P1052 השני; V397 אחר<br> | ||
| + | והמספר האחר: Mo30 והשני הוא</ref> | ||
|- | |- | ||
| | | | ||
::The decade is 300, its square is 90 thousand. We subtract from it the square of 50, which is the deficit and the excess, and it is 2500. The remainder is the sought after. | ::The decade is 300, its square is 90 thousand. We subtract from it the square of 50, which is the deficit and the excess, and it is 2500. The remainder is the sought after. | ||
| − | |style="text-align:right;"|והנה הכלל<ref group=I>והנה הכלל: Mo30 והמספר כלל< | + | |style="text-align:right;"|והנה הכלל הוא ש' ומרובעו צ' אלף נחסר ממנו מרובע נ' שהוא החסרון והיתרון ומספרו אלפים ות"ק והנשאר הוא המבוקש‫<ref group=I>והנה הכלל: Mo30 והמספר כלל<br> |
| + | הוא: Lo27153; P1051 om.<br> | ||
| + | ומרובעו: Mu150 והמרובע; P1029 ומרובעו הוא; P1052 והמרובע הוא<br> | ||
| + | צ‫': P1029 <s>ה</s> צ‫'<br> | ||
| + | מרובע: Lo27153 om.<br> | ||
| + | שהוא: Mu150 והוא מרובע; O187; P1029; P1050; P1052; V398 שהוא מרובע; Lo27153 על חמשים שהוא מרובע; P1051 שהוא המרובע<br> | ||
| + | החסרון והיתרון: Mo30 היתרון והחסרון<br> | ||
| + | ומספרו: Mo30 ומרובעו<br> | ||
| + | המבוקש: O187 המבוקש פ"ז אלף ות"ק; Lo27153 המבוקש שהוא פ"ז אלפי' וחמש מאות; P1052 המבוקש marg. והנה המבוקש פ"ז אלף ת"ק</ref> | ||
|- | |- | ||
| colspan=2| | | colspan=2| | ||
| Line 372: | Line 850: | ||
| | | | ||
:According to this procedure we can proceed with other numbers that are similar to these, such that the deficit is the same as the excess. | :According to this procedure we can proceed with other numbers that are similar to these, such that the deficit is the same as the excess. | ||
| − | |style="text-align:right;"|ועל זה הסדר<ref group=I>הסדר: O187; P1052; V398; W152 הדרך; P1051 הדרך והסדר<br>זה הסדר: P1029 סדר זה< | + | |style="text-align:right;"|ועל זה הסדר נוכל לעשות שאר המספרים הדומים לאלה שהחסרון כמו היתרון‫<ref group=I>הסדר: O187; P1052; V398; W152 הדרך; P1051 הדרך והסדר<br> |
| + | זה הסדר: P1029 סדר זה<br> | ||
| + | נוכל: Mu150; P1052 תוכל<br> | ||
| + | שאר: P1051 om.<br> | ||
| + | המספרים: P1029; P1052 מספרים<br> | ||
| + | לאלה: Mo30; Mu150 לאלו<br> | ||
| + | שהחסרון: Mu150 כשיהיה החסרון; V397 כי יהיה ה<s>א</s><sup>ח</sup>סרון; III; P1051; P1052; V398 כי החסרון<br> | ||
| + | כמו היתרון: Mu150 כמו היתרון וזה צורתו; O187 והיתרון שוים</ref> | ||
|- | |- | ||
| | | | ||
| Line 380: | Line 865: | ||
| | | | ||
:Another important method that I have found is with thirds: We take one-third of the number and we know how much its square is. We take its closest higher decade and subtract from it the square of the third; the remainder is the sought after. | :Another important method that I have found is with thirds: We take one-third of the number and we know how much its square is. We take its closest higher decade and subtract from it the square of the third; the remainder is the sought after. | ||
| − | |style="text-align:right;" | + | |style="text-align:right;"|‫<ref group=note>W152 marg. לחזור על הטעם של [הטענה] דרך כזה</ref>'''דרך אחרת''' נכבדת שהוצאתי בדרך השלישית שנקח שלישית החשבון ונדע כמה מרובעו <div id="higher decade"></div>ונקח כמוהו בכלל הגבוה‫<ref group=note>[[Excerpts_Attributed_to_Immanuel_Bonfils#Higher_decade|<span style=color:blue>commentary: higher decade</span>]]</ref> ממנו ונחסר מרובע השלישית ממנו <div id="the remainder is the required"></div>והנשאר הוא המבוקש‫<ref group=note>[[Excerpts_Attributed_to_Immanuel_Bonfils#The_remainder_is_the_required|<span style=color:blue>commentary: the remainder is the required</span>]]</ref> |
| + | <ref group=I>נכבדת: P1052 מצאתי נכבדת<br> | ||
| + | שהוצאתי: Mo30 הוצאתיה; Mu150 מאצתי שהוצאתי marg. מצאתי במרובעים<br> | ||
| + | בדרך: O187 מן<br> | ||
| + | השלישית: P1050 השלישיות<br> | ||
| + | שנקח: Mo30 והוא שנקח; P1051 שהוא<br> | ||
| + | החשבון: Mo30 om.<br> | ||
| + | מרובע השלישית ממנו: O187 ממנו מרובע השלישית</ref> | ||
|- | |- | ||
| | | | ||
:*{{#annot:3²|857|GMCO}}Example: we wish to know how much is the square number of 3. | :*{{#annot:3²|857|GMCO}}Example: we wish to know how much is the square number of 3. | ||
::<math>\scriptstyle3^2</math> | ::<math>\scriptstyle3^2</math> | ||
| − | |style="text-align:right;"|‫<ref group=I>O187 example is missing< | + | |style="text-align:right;"|<big>דמיון</big> בקשנו לדעת כמה מספר מרובע ג‫'{{#annotend:GMCO}}‫<ref group=I>O187 example is missing<br> |
| + | בקשנו: Mo30 רצינו<br> | ||
| + | מספר מרובע: W152 מרובע מספר</ref> | ||
|- | |- | ||
| | | | ||
::We take its third, which is one; its square is one; ten is its closest decade. We subtract from it the square of one, which is the third; 9 remain and this is the sought after. | ::We take its third, which is one; its square is one; ten is its closest decade. We subtract from it the square of one, which is the third; 9 remain and this is the sought after. | ||
| − | |style="text-align:right;"|נקח<ref group=I>נקח: Mu150 ונקח<br>ג' נקח: W152 top< | + | |style="text-align:right;"|נקח שלישיתו שהוא אחד ומרובעו אחד ועשרה שהוא הכלל הקרוב אליו נחסר ממנו מרובע אחד שהוא השלישית וישאר ט' והוא המבוקש‫<ref group=I>נקח: Mu150 ונקח<br> |
| + | ג' נקח: W152 top<br> | ||
| + | שלישיתו: P1051; V398; W194 שלישית; W152 השלישית<br> | ||
| + | שהוא: P1051 והוא<br> | ||
| + | ומרובעו: P1029 ומרובעו שהוא<br> | ||
| + | אחד: Lo27153 <s>אחד</s> אחד; Mu150 הוא א‫'<br> | ||
| + | ומרובעו אחד: P1051 om.<br> | ||
| + | ועשרה: Mo30; Mu150; P1029; P1050; P1051; V398; III והוא עשרה; P1029 marg. והנה<br> | ||
| + | הכלל: P1050 כלל<br> | ||
| + | שהוא הכלל: Mo30 בכלל<br> | ||
| + | ממנו: V398 om.<br> | ||
| + | מרובע אחד שהוא: Mo30 מרובע אחד שהוא אחד; Lo27153 מרובע אחד שהוא <s>עשרה</s> א‫'<br> | ||
| + | השלישית: Mo30; Lo27153 om.<br> | ||
| + | וישאר: Mo30 והנשאר<br> | ||
| + | ט' והוא: Mo30 שהוא ט' הוא</ref> | ||
|- | |- | ||
| colspan=2| | | colspan=2| | ||
| Line 397: | Line 905: | ||
:*{{#annot:15²|857|FZlV}}Another example: we wish to know the square of 15. | :*{{#annot:15²|857|FZlV}}Another example: we wish to know the square of 15. | ||
::<math>\scriptstyle15^2</math> | ::<math>\scriptstyle15^2</math> | ||
| − | |style="text-align:right;"|<big>דמיון אחר</big><ref group=I>אחר: O187 om.; Lo27153 <s>אחר</s> אחר<br>דמיון אחר: P1050 ד"א</ref> | + | |style="text-align:right;"|<big>דמיון אחר</big> בקשנו לדעת מרובע ט"ו{{#annotend:FZlV}}‫<ref group=I>אחר: O187 om.; Lo27153 <s>אחר</s> אחר<br> |
| + | דמיון אחר: P1050 ד"א</ref> | ||
|- | |- | ||
| | | | ||
::Its one-third is 5, the square of which is 25, the analogous number in the closest decade is 250. Subtract from it the square of 5, which is the third; 225 remain. | ::Its one-third is 5, the square of which is 25, the analogous number in the closest decade is 250. Subtract from it the square of 5, which is the third; 225 remain. | ||
| − | |style="text-align:right;"|ושלישיתו ה' ומרובעו כ"ה והדומה<ref group=I>והדומה: Mo30; O187 והדומה לו< | + | |style="text-align:right;"|ושלישיתו ה' ומרובעו כ"ה והדומה בכלל הקרוב אליו ר"נ חסר ממנו מרובע ה' שהוא השלישית ישאר רכ"ה‫<ref group=I>והדומה: Mo30; O187 והדומה לו<br> |
| + | אליו: Mo30; V398; P1050; III om.<br> | ||
| + | ר"נ: Mo30; Mu150 הוא ר"נ<br> | ||
| + | חסר: Mu150 נחסר<br> | ||
| + | ה' שהוא השלישית: Mu150 שלישי שהוא כ"ה<br> | ||
| + | ישאר: O187 וישאר; V397; V398 נשאר<br> | ||
| + | רכ"ה: Mo30 רכ"ה והוא המבוקש; Mu150 רכ"ה על צורה זו ה'ב'ב' והוא המבוקש</ref> | ||
|- | |- | ||
| colspan=2| | | colspan=2| | ||
| Line 409: | Line 924: | ||
:*{{#annot:24²|857|Ny3j}}Another example: we wish to know how much is the square of 24. | :*{{#annot:24²|857|Ny3j}}Another example: we wish to know how much is the square of 24. | ||
::<math>\scriptstyle24^2</math> | ::<math>\scriptstyle24^2</math> | ||
| − | |style="text-align:right;"|‫<ref group=I>III example is missing< | + | |style="text-align:right;"|<big>דמיון אחר</big> בקשנו לדעת כמה מרובע כ"ד{{#annotend:Ny3j}}‫<ref group=I>III example is missing<br> |
| + | דמיון אחר: P1050 ד"א<br> | ||
| + | כמה: Mo30 om.</ref> | ||
|- | |- | ||
| | | | ||
::Its one-third is 8, the square of which is 64 and its analogous number in the closest higher rank is 640. We subtract from it the square of the third, which is 64; 576 remain and this is the sought after. | ::Its one-third is 8, the square of which is 64 and its analogous number in the closest higher rank is 640. We subtract from it the square of the third, which is 64; 576 remain and this is the sought after. | ||
| − | |style="text-align:right;"|הנה<ref group=I>הנה: P1029; P1051 om.< | + | |style="text-align:right;"|הנה שלישיתו ח' ומרובעו ס"ד ודמיונו במעלה הגבוהה ממנו תר"מ נחסר ממנו מרובע השלישית שהוא ס"ד ישאר תקע"ו והוא המבוקש‫<ref group=I>הנה: P1029; P1051 om.<br> |
| + | הנה שלישיתו: Mo30 ושלישיתו<br> | ||
| + | ודמיונו: P1051; V397 ודמיון<br> | ||
| + | הגבוהה: O187; P1029; P1050 הגבוה; P1051 העליונה; V397 <sup>ה</sup>גבוה<br> | ||
| + | במעלה הגבוהה: Mo30 בכלל הגבוה<br> | ||
| + | תר"מ: Mo30 הוא תר"מ<br> | ||
| + | ממנו: P1050; P1051; V398 om.<br> | ||
| + | מרובע: V397 om.<br> | ||
| + | ישאר: P1050 וישאר; V397 נשארו</ref> | ||
|- | |- | ||
| colspan=2| | | colspan=2| | ||
| Line 424: | Line 949: | ||
| | | | ||
:If the number does not have a whole third and there is an excess of one, subtract the one from the number and calculate the sought number in the procedure that I have shown you. Add to the result the number that has a [whole] third and the [original] number itself, so the sum is the sought after. | :If the number does not have a whole third and there is an excess of one, subtract the one from the number and calculate the sought number in the procedure that I have shown you. Add to the result the number that has a [whole] third and the [original] number itself, so the sum is the sought after. | ||
| − | |style="text-align:right;"|ואם לא היה<ref group= | + | |style="text-align:right;"|ואם לא היה למספר שלישית שלמה ויהיה בו תוספת אחד<br> |
| − | + | חסר האחד מהמספר והוצא המספר המבוקש כמשפט שהראיתיך ומה שיעלה הוסף עליו המספר שיש לו שלישית והמספר בעצמו <div id="the sum is the required"></div>והמחובר הוא המבוקש‫<ref group=note>[[Excerpts_Attributed_to_Immanuel_Bonfils#The_sum_is_the_required|<span style=color:blue>commentary: the sum is the required</span>]]</ref><ref group=I>היה: Mo30; O187 יהיה<br> | |
| + | בו: P1051; V397 om.<br> | ||
| + | אחד: O187 של אחד<br> | ||
| + | חסר: P1051 נחסר<br> | ||
| + | האחד: Mo30; O187; P1051 אחד<br> | ||
| + | מהמספר: O187 מן המספר<br> | ||
| + | והוצא: Mo30 ותוציא<br> | ||
| + | המספר: III om.<br> | ||
| + | כמשפט: Mo30 כמו<br> | ||
| + | שיעלה: O187 שיעלה לך<br> | ||
| + | הוסף: P1050 הוצא<br> | ||
| + | שיש לו: P1029 twice<br> | ||
| + | שלישית: Mo30 ב' שלישית</ref> | ||
|- | |- | ||
| | | | ||
| Line 434: | Line 971: | ||
| | | | ||
::It has no third, so we subtract from it one, which is the excess, one-third of the remainder is two, the square of which is four and the closest analogous decade is 40. We subtract from it 4, which is the square of the third; 36 remain; which is the square of 6. We add to it the 6, which has a third, and the 7 that was our number originally, both together are 13, the sum is 49, and this is the square of 7. | ::It has no third, so we subtract from it one, which is the excess, one-third of the remainder is two, the square of which is four and the closest analogous decade is 40. We subtract from it 4, which is the square of the third; 36 remain; which is the square of 6. We add to it the 6, which has a third, and the 7 that was our number originally, both together are 13, the sum is 49, and this is the square of 7. | ||
| − | |style="text-align:right;"|והנה אין<ref group=I>אין: V398 om.< | + | |style="text-align:right;"|והנה אין לו שלישית חסרנו ממנו אחד שהוא נוסף והנה שלישית הנשאר שנים ומרובעו ארבעה והנה בכלל הקרוב הדומה אליו מ' נחסר ממנו ד' שהוא מרובע השלישית וישאר ל"ו שהוא מרובע ו' נחבר אליו הו' שיש לו שלישית והז' שהיה מספרנו בראשונה ושניהם י"ג יהיה המחובר מ"ט והוא מרובע ז‫'<ref group=I>אין: V398 om.<br> |
| + | לו: V398 <s>לו</s> marg. לו<br> | ||
| + | חסרנו: O187 חסר<br> | ||
| + | ממנו: Mo30 om.<br> | ||
| + | נוסף: V398 marg.<br> | ||
| + | שלישית: O187 שלישיתו; P1029 השלישית<br> | ||
| + | והנה שלישית: Mo30 ושלישית<br> | ||
| + | הנשאר: O187 om.<br> | ||
| + | והנה: Mo30 והוא<br> | ||
| + | הדומה: Mo30; III om.<br> | ||
| + | מ': P1029 om.<br> | ||
| + | נחסר: P1051 <s>נסר</s> נחסר<br> | ||
| + | ממנו: Mo30 om.<br> | ||
| + | השלישית: Mo30 שלישית<br> | ||
| + | וישאר: Mo30; P1029 ישאר; V397 ונשאר; W152 וישארו<br> | ||
| + | שהוא: Mo30 והוא<br> | ||
| + | אליו: P1029; P1050; P1051; V398 עליו<br> | ||
| + | שלישית: O187 השלישית<br> | ||
| + | שהיה: P1051 שיהיה; V397 שהוא<br> | ||
| + | המחובר: III המרובע</ref> | ||
|- | |- | ||
| colspan=2| | | colspan=2| | ||
| Line 442: | Line 998: | ||
:*{{#annot:22²|857|tUlx}}Another example: we wish to know how much is the square of 22. | :*{{#annot:22²|857|tUlx}}Another example: we wish to know how much is the square of 22. | ||
::<math>\scriptstyle22^2</math> | ::<math>\scriptstyle22^2</math> | ||
| − | |style="text-align:right;"|<big>דמיון אחר</big><ref group=I>דמיון אחר: P1050 ד"א< | + | |style="text-align:right;"|<big>דמיון אחר</big> רצינו לדעת כמה מרובע כ"ב{{#annotend:tUlx}}‫<ref group=I>דמיון אחר: P1050 ד"א<br> |
| + | רצינו: V397 בקשנו<br> | ||
| + | לדעת: O187 לידע<br> | ||
| + | כמה: Mo30 om.</ref> | ||
|- | |- | ||
| | | | ||
::We subtract one; 21 remain, its third is 7, the square of which is 49 and its closest decade is 490. We subtract from it 49, which is the square of the third; 441 remain, which is the square of 21. We add 21 as well as 22 together, which are 43; the sum is 484 and this is the square of 22. | ::We subtract one; 21 remain, its third is 7, the square of which is 49 and its closest decade is 490. We subtract from it 49, which is the square of the third; 441 remain, which is the square of 21. We add 21 as well as 22 together, which are 43; the sum is 484 and this is the square of 22. | ||
| − | |style="text-align:right;"|והנה<ref group=I>והנה: Mo30 om.< | + | |style="text-align:right;"|והנה חסרנו אחד ונשאר כ"א ושלישיתו ז' ומרובעו מ"ט והנה בכלל הקרוב אליו ת"צ נחסר ממנו מ"ט שהוא מרובע השלישית נשארו תמ"א שהוא מרובע כ"א נוסיף כ"א גם כ"ב מחוברים שהם מ"ג יעלה המחובר תפ"ד וזהו מרובע כ"ב‫<ref group=I>והנה: Mo30 om.<br> |
| + | ונשאר: P1029 נשאר<br> | ||
| + | ושלישיתו: O187 ושלישית כ"א<br> | ||
| + | ומרובעו: O187 <s>ומרובעו</s> ומרובעו<br> | ||
| + | והנה: Mo30 ויהיה; O187 והנה יהיה<br> | ||
| + | בכלל: Mo30; O187 הכלל<br> | ||
| + | הקרוב: Mo30 הגבוה הגבוה; O187 הקרוב הדומה<br> | ||
| + | אליו: P1050 om.<br> | ||
| + | ת"צ: P1029; P1050; P1051; V397; V398; Lo27153; W194 ד' מאות וצ‫'<br> | ||
| + | ממנו: P1051 om.<br> | ||
| + | השלישית: V397 השלישי<br> | ||
| + | שהוא מרובע השלישית: Mo30; III om.<br> | ||
| + | נשארו: V397 marg.; W152 ישארו<br> | ||
| + | גם: Mo30; O187 עם<br> | ||
| + | מחוברים: O187 מחבורים; P1051 מחוברין<br> | ||
| + | שהם: Mo30 ויעלה<br> | ||
| + | מחוברים שהם מ"ג: III om.<br> | ||
| + | יעלה: Mo30 ויעלה<br> | ||
| + | המחובר: Mo30 המבוקש; O187 מחבורם; P1051; V397 המספר<br> | ||
| + | וזהו: Mo30 והוא; O187; P1029 וזה; W152 וזה הוא</ref> | ||
|- | |- | ||
| colspan=2| | | colspan=2| | ||
| Line 457: | Line 1,035: | ||
| | | | ||
:If there are 2 between our number and the number that has a third, we do the opposite by adding one to our number and know how much is the square of the number that has a third. We subtract from it the number that has a third and the number that we had, and the remainder is the sought after. | :If there are 2 between our number and the number that has a third, we do the opposite by adding one to our number and know how much is the square of the number that has a third. We subtract from it the number that has a third and the number that we had, and the remainder is the sought after. | ||
| − | |style="text-align:right;"|ואם היו<ref group=I>היו: O187 יהיה; P1051; V397 יהיו< | + | |style="text-align:right;"|ואם היו שנים בין המספר שלנו ובין המספר שיש לו שלישית<br> |
| − | + | נעשה להפך שנוסיף על המספר שלנו אחד ונדע כמה מרובע מספר שיש לו שלישית ונחסר ממנו כמספר שיש לו שלישית וכמספר שהיה לנו והנשאר הוא המבוקש‫<ref group=I>היו: O187 יהיה; P1051; V397 יהיו<br> | |
| + | שנים: P1051 תוספת שנים<br> | ||
| + | המספר: P1050 המספר<s>ים</s>; P1029; V398 המספרים<br> | ||
| + | שלנו: P1051 שיש לנו<br> | ||
| + | ובין: P1050 top<br> | ||
| + | ובין המספר: P1051 למספר הנקדם; V398; Lo27153; P1029; W194 ומספר<br> | ||
| + | שלישית: P1051 שלישית מהמספר הנקדם בזה הדרך והוא שנקח מהכל שלישיתו ונקח כמוהו בכלל הגבוה ממנו ונחסר<br> | ||
| + | שנוסיף: P1051 שהוסיף<br> | ||
| + | שלנו: O187 om.<br> | ||
| + | מרובע: III מחובר; P1050 <s>ה</s>מרובע<br> | ||
| + | מספר: P1050 <sup>ה</sup>מספר; P1051 ממספר זה; III מספר אחר<br> | ||
| + | שלישית: P1051 שלישית עתה בתוספת האחד ממנו ומרובע השלישית<br> | ||
| + | שיש לו שלישית: P1050 שיש <sup>לו</sup> שלישית <s>שיש לו ש</s><br> | ||
| + | נעשה להפך ... שיש לו שלישית: Mo30 twice<br> | ||
| + | ונחסר: Mo30 וכמספר שהיה לנו ונחסר; P1051 עוד נחסר<br> | ||
| + | כמספר: Lo27153 כמספר marg. המספר<br> | ||
| + | ונחסר ממנו כמספר שיש לו שלישית: V397 om.<br> | ||
| + | וכמספר: P1050 ג"כ כמספר; V398; III גם המספר</ref> | ||
|- | |- | ||
| | | | ||
:*{{#annot:23²|857|eFIa}}Example: we wish to know how much is the square of 23. | :*{{#annot:23²|857|eFIa}}Example: we wish to know how much is the square of 23. | ||
::<math>\scriptstyle23^2</math> | ::<math>\scriptstyle23^2</math> | ||
| − | |style="text-align:right;"|<big>דמיון</big> בקשנו לדעת כמה מרובע<ref group=I>מרובע: Lo27153 מחובר</ref> | + | |style="text-align:right;"|<big>דמיון</big> בקשנו לדעת כמה מרובע כ"ג{{#annotend:eFIa}}‫<ref group=I>מרובע: Lo27153 מחובר</ref> |
|- | |- | ||
| | | | ||
::Since it does not have a whole third, we add one; it is 24, its third is 8, the square of which is 64 and the analogous number is 640. We subtract from it 64, which is the square of the third; 576 remain, which is the square of 24. We subtract from this number 47, which is the sum of 24 with 23; 529 remain and this is the sought after. | ::Since it does not have a whole third, we add one; it is 24, its third is 8, the square of which is 64 and the analogous number is 640. We subtract from it 64, which is the square of the third; 576 remain, which is the square of 24. We subtract from this number 47, which is the sum of 24 with 23; 529 remain and this is the sought after. | ||
| − | |style="text-align:right;"|והנה בעבור שאין לו<ref group=I>לו: Lo27153; Mo30 om.< | + | |style="text-align:right;"|והנה בעבור שאין לו שלישית שלמה נוסיף אחד יהיו כ"ד ושלישיתו ח' ומרובעו ס"ד והדומה לו תר"מ נחסר ממנו ס"ד שהוא מרובע השלישית ישאר תקע"ו והוא מרובע כ"ד גם נחסר מזה המספר מ"ז שהוא כ"ד עם כ"ג מחוברים ויהיה הנשאר תקכ"ט והוא המבוקש‫<ref group=I>לו: Lo27153; Mo30 om.<br> |
| + | לו שלישית שלמה: P1029 שלישית שלימה לו<br> | ||
| + | יהיו: Lo27153 יהיה; Mo30; P1029; W152 ויהיו; P1051 ויהיה<br> | ||
| + | ושלישיתו: P1051 השלישיתו<br> | ||
| + | לו: V397 om.<br> | ||
| + | ישאר: P1050 וישאר; W152 ישארו<br> | ||
| + | גם: Mo30 om.<br> | ||
| + | גם נחסר: III נחסר גם<br> | ||
| + | המספר: O187 מספר<br> | ||
| + | מזה המספר: Mo30 ממנו; P1029 ממספר זה<br> | ||
| + | מחוברים: P1050; P1051; V398; III מחוברין<br> | ||
| + | מ"ז ... מחוברים: Mo30 כ"ד וכ"ג ויעלה מ"ז; O187 כ"ד עם כ"ג ויעלה מ"ז<br> | ||
| + | ויהיה הנשאר: Mo30 והנשאר; O187 ישאר; V397 ונשאר</ref> | ||
|- | |- | ||
| colspan=2| | | colspan=2| | ||
| Line 481: | Line 1,088: | ||
| | | | ||
:Know that if there are two digits to multiply by one another, once will be enough for you. | :Know that if there are two digits to multiply by one another, once will be enough for you. | ||
| − | |style="text-align:right;"|ודע כי אם יהיו<ref group=I>יהיו: Mo30; P1029 היו; O187; P1051 יהיו לך< | + | |style="text-align:right;"|<div id="one_digit_by_one_digit"></div>ודע כי אם יהיו שנים מספרים לכפול זה על זה יספיק לך פעם אחת‫<ref group=note>[[Excerpts_Attributed_to_Immanuel_Bonfils#Know_that_if_there_are_two_digits_to_multiply|<span style=color:blue>commentary: know that if there are two digits to multiply</span>]]</ref> |
| + | <ref group=I>יהיו: Mo30; P1029 היו; O187; P1051 יהיו לך<br> | ||
| + | שנים: O187 שני</ref> | ||
|- | |- | ||
| | | | ||
| Line 489: | Line 1,098: | ||
| | | | ||
:If one digit by two digits, you have to do this twice. | :If one digit by two digits, you have to do this twice. | ||
| − | |style="text-align:right;"|ואם היה<ref group=I>היה: Mo30; O187 היה לך; V397 יהיה< | + | |style="text-align:right;"|<div id="two_digits_by_two_digits"></div>ואם היה מספר אחד על שנים מספרים אתה צריך לעשות זה פעמים‫<ref group=note>[[Excerpts_Attributed_to_Immanuel_Bonfils#You_have_to|<span style=color:blue>commentary: you have to</span>]]</ref> |
| + | <ref group=I>היה: Mo30; O187 היה לך; V397 יהיה<br> | ||
| + | אחד: W152 אחת<br> | ||
| + | על: Lo27153 <sup>על</sup> <s>על</s><br> | ||
| + | שנים: O187 שני<br> | ||
| + | מספרים: Mo30 om.<br> | ||
| + | פעמים: O187; P1029 שני פעמים</ref> | ||
|- | |- | ||
| | | | ||
| Line 497: | Line 1,112: | ||
| | | | ||
:If by three [digits], three times. | :If by three [digits], three times. | ||
| − | |style="text-align:right;"|ואם על שלשה שלשה‫<ref group=I>על שלשה שלשה: Lo27153 <s>ג' על ג'</s> על ג' ג'</ref> | + | |style="text-align:right;"|ואם על שלשה שלשה‫<ref group=I>על שלשה שלשה: Lo27153 <s>ג' על ג'</s> על ג' ג‫'</ref> |
|- | |- | ||
|And so on according to this rule. | |And so on according to this rule. | ||
| − | |style="text-align:right;"|ועל<ref group=I>ועל: V398; P1050; III על</ref> | + | |style="text-align:right;"|ועל זה המשפט הכל‫<ref group=I>ועל: V398; P1050; III על</ref> |
|- | |- | ||
| | | | ||
| Line 508: | Line 1,123: | ||
| | | | ||
:If they are two numbers by two numbers, you have to do this 4 times. | :If they are two numbers by two numbers, you have to do this 4 times. | ||
| − | |style="text-align:right;"|ואם הם שני<ref group=I>שני: P1050; P1051 שנים< | + | |style="text-align:right;"|ואם הם שני מספרים על שני מספרים אתה צריך לעשות זה ד' פעמים‫<ref group=I>שני: P1050; P1051 שנים<br> |
| + | שני: Lo27153 <s>ב'</s> ב'; P1050 שנים<br> | ||
| + | על שני מספרים: P1051 om.<br> | ||
| + | אתה צריך: P1029 צריך אתה<br> | ||
| + | לעשות: P1051 om.<br> | ||
| + | זה: W152 top<br> | ||
| + | לעשות זה: V397 זה לעשות</ref> | ||
|- | |- | ||
| | | | ||
| Line 517: | Line 1,138: | ||
| | | | ||
::We multiply 10 by 20, which is a decade, also 10 by 8; the result is 280. Then we multiply 3 by 20 and by 8; the result is 84. So the total is 364. | ::We multiply 10 by 20, which is a decade, also 10 by 8; the result is 280. Then we multiply 3 by 20 and by 8; the result is 84. So the total is 364. | ||
| − | |style="text-align:right;"|והנה<ref group=I>והנה: O187; V398; P1029; P1050; III הנה< | + | |style="text-align:right;"|והנה כפלנו י' על כ' שהוא כלל גם י' על ח' עלו ר"פ ואחר כפלנו ג' על כ' גם על ח' עלו פ"ד והנה הכל שס"ד‫<ref group=I>והנה: O187; V398; P1029; P1050; III הנה<br> |
| + | שהוא כלל: P1050 שהוא כלל <sup>עלו ר‫'</sup><br> | ||
| + | י‫': Mo30; P1029; P1050; P1051; V397; III om.<br> | ||
| + | גם י‫': O187 om.<br> | ||
| + | על: O187 ועל<br> | ||
| + | ח‫': P1029 הח‫'<br> | ||
| + | עלו: Mo30 ויעלו; V398 om.<br> | ||
| + | ר"פ: V397 <s>פ"ד</s> <sup>ר"פ</sup>; V398 marg.<br> | ||
| + | ואחר: Mo30; P1050; III גם; O187; P1029; P1051; V398 וגם<br> | ||
| + | גם: O187 ג‫'; P1051 וגם<br> | ||
| + | גם על: Mo30 ועל<br> | ||
| + | עלו: Mo30 ויעלו<br> | ||
| + | ואחר ... פ"ד: V397 om.<br> | ||
| + | והנה: Mo30 ויהיה<br> | ||
| + | הכל: V397 הכלל</ref> | ||
|- | |- | ||
| colspan=2| | | colspan=2| | ||
| Line 528: | Line 1,163: | ||
| | | | ||
:If there is one decade shared by the two numbers, three times are enough for you. | :If there is one decade shared by the two numbers, three times are enough for you. | ||
| − | |style="text-align:right;"|ואם היה כלל אחד כולל<ref group=I>כולל: P1029 נופל< | + | |style="text-align:right;"|ואם היה כלל אחד כולל שני המספרים די לך בג' פעמים‫<ref group=I>כולל: P1029 נופל<br> |
| + | שני: P1029 בשני<br> | ||
| + | המספרים: Mo30; P1051; V397 מספרים; P1029 <sup>ה</sup>מספרים<br> | ||
| + | לך: O187 om.</ref> | ||
|- | |- | ||
| | | | ||
:*{{#annot:13×16|156|2VDH}}Example: we wish to multiply 13 by 16. | :*{{#annot:13×16|156|2VDH}}Example: we wish to multiply 13 by 16. | ||
::<math>\scriptstyle13\times16</math> | ::<math>\scriptstyle13\times16</math> | ||
| − | |style="text-align:right;"|<big>דמיון</big> בקשנו<ref group=I>בקשנו: V398 רצינו< | + | |style="text-align:right;"|<big>דמיון</big> בקשנו לכפול י"ג על י"ו{{#annotend:2VDH}}‫<ref group=I>בקשנו: V398 רצינו<br> |
| + | לכפול: P1051 om.; V397 לדעת</ref> | ||
|- | |- | ||
| | | | ||
::10 is shared by the two numbers. We add 3 with 6 and with 10, so our number is 19. We multiply it by ten; the result is 190. We multiply the two small numbers, which are 3 by 6; the result is 18. So the total is 208. | ::10 is shared by the two numbers. We add 3 with 6 and with 10, so our number is 19. We multiply it by ten; the result is 190. We multiply the two small numbers, which are 3 by 6; the result is 18. So the total is 208. | ||
| − | |style="text-align:right;"|והנה<ref group=I>והנה: P1050 הנה< | + | |style="text-align:right;"|והנה י' כולל שני המספרים והנה נחבר ג' עם ו' עם י' והנה יהיה מספרינו י"ט נכפול אותו בעשרה עלו ק"צ נכפול שני המספרים הקטנים שהם ג' על ו' יעלו י"ח והנה הכל ר"ח‫<ref group=I>והנה: P1050 הנה<br> |
| + | שני: P1051; V397 om.; P1050 top<br> | ||
| + | המספרים: Mo30 מספרים<br> | ||
| + | נחבר: O187; P1029; P1050; P1051; V397; III חברנו<br> | ||
| + | עם: Mo30 על<br> | ||
| + | ו‫': P1029 ו' ועלו ט‫'<br> | ||
| + | עם: O187; P1050 ועם; V398; III על; Lo27153 <sup>גם</sup> על<br> | ||
| + | עם י‫': Mo30 ויהיו י"ט; P1029 om.<br> | ||
| + | ו' עם י‫': P1051 י'ו‫'<br> | ||
| + | והנה יהיה: O187 ויהיה<br> | ||
| + | נכפול: Mo30; P1051; III וכפול; P1029 וכפלנו<br> | ||
| + | אותו: V397; W152 אותה; P1050; P1051; Lo27153; W194 אותם; P1029 om.<br> | ||
| + | עלו: Mo30 ויעלה<br> | ||
| + | ק"צ: Lo27153 <s>ק"ץ</s> ק"צ<br> | ||
| + | נכפול: Lo27153 וכפול; O187 ונכפול<br> | ||
| + | שני: P1051; V397 שנים; P1029 לשני<br> | ||
| + | הקטנים: Lo27153 om.<br> | ||
| + | שני המספרים הקטנים שהם: Mo30 om.<br> | ||
| + | יעלו: Mo30 ויעלה; P1050; V398; III עלו<br> | ||
| + | והנה הכל: O187 והכל<br> | ||
| + | ר"ח: V398 כ"ח marg. ר"ח<br> | ||
| + | והנה הכל ר"ח: Mo30 ונשים אותם על ק"צ <sup>ויעלה ר"ח</sup> והוא המבוקש</ref> | ||
|- | |- | ||
| colspan=2| | | colspan=2| | ||
| Line 544: | Line 1,204: | ||
| | | | ||
:Sometimes twice alone are enough for you. | :Sometimes twice alone are enough for you. | ||
| − | |style="text-align:right;"|ויש<ref group=I>ויש: V398; P1050; III והנה יש; P1029 וזה< | + | |style="text-align:right;"|ויש שיספיק לך שני פעמים לבדם‫<ref group=I>ויש: V398; P1050; III והנה יש; P1029 וזה<br> |
| + | שני: P1029; P1050; P1051 om.<br> | ||
| + | לך שני: O187 om.<br> | ||
| + | שיספיק לך שני: III לך פעמים שיספיק</ref> | ||
|- | |- | ||
| | | | ||
:*{{#annot:24×26|156|HqY7}}Example: we wish to multiply 24 by 26. | :*{{#annot:24×26|156|HqY7}}Example: we wish to multiply 24 by 26. | ||
::<math>\scriptstyle24\times26</math> | ::<math>\scriptstyle24\times26</math> | ||
| − | |style="text-align:right;"|<big>דמיון</big><ref group=I>דמיון: V397 דמיון בקשנו לדעת י"ג על י"ו והנה י' כולל המספרים והנה חברנו ג' עם ו' דמיון< | + | |style="text-align:right;"|<big>דמיון</big> בקשנו לכפול כ"ד על כ"ו{{#annotend:HqY7}}‫<ref group=I>דמיון: V397 דמיון בקשנו לדעת י"ג על י"ו והנה י' כולל המספרים והנה חברנו ג' עם ו' דמיון<br> |
| + | לכפול: P1029 top<br> | ||
| + | כ"ד: O187; P1029; P1050; P1051; W152; W194 חשבון כ"ד<br> | ||
| + | כ"ו: O187; P1029; P1051 חשבון כ"ו</ref> | ||
|- | |- | ||
| | | | ||
::20 is shared by the two numbers. We add 4 with 26, which is the greater number; the sum is 30. We multiply 20 by 30; the result is 600. We multiply the smaller [numbers] by each other; the result is 24. So the sought for is 624. | ::20 is shared by the two numbers. We add 4 with 26, which is the greater number; the sum is 30. We multiply 20 by 30; the result is 600. We multiply the smaller [numbers] by each other; the result is 24. So the sought for is 624. | ||
| − | |style="text-align:right;"|הנה<ref group=I>הנה: V397 והנה< | + | |style="text-align:right;"|הנה כ' כולל שני המספרים חברנו ד' עם כ"ו שהוא הגדול עלה המספר ל' כפלנו כ' על ל' עלו ת"ר וכפלנו הקטנים זה על זה עלו כ"ד והנה המבוקש תרכ"ד‫<ref group=I>הנה: V397 והנה<br> |
| + | כ‫': W152 הכ‫'<br> | ||
| + | שני: P1050; P1051; V397; V398 om.; P1029 top<br> | ||
| + | עם: O187; P1029; P1050; P1051; V398; III על<br> | ||
| + | שהוא הגדול: Mo30 om.; V397 marg. שהוא המספר הגדול<br> | ||
| + | עלה: P1051; V397 עולה; P1029 om.<br> | ||
| + | עלו: Mo30 עלה<br> | ||
| + | וכפלנו: Mo30 כפלנו<br> | ||
| + | עלו: Mo30 יהיה; O187 ועלו<br> | ||
| + | עלו כ"ד: P1050 marg.<br> | ||
| + | והנה: Mo30 והיה; O187 הנה<br> | ||
| + | המבוקש: III המחובר; P1029 המבוקר<sup>ש</sup><br> | ||
| + | ויש שיספיק לך ... תרכ"ד: Mo30 marg.</ref> | ||
|- | |- | ||
| colspan=2| | | colspan=2| | ||
| Line 564: | Line 1,242: | ||
| | | | ||
:If you multiply 3 digits by 3 [digits], you have to do this nine times. | :If you multiply 3 digits by 3 [digits], you have to do this nine times. | ||
| − | |style="text-align:right;"|ואם | + | |style="text-align:right;"|ואם תכפול ג' מספרים על ג' אתה צריך לעשות זה ט' פעמים‫<ref group=I>ואם: W152 אם<br> |
| + | ג‫': O187; III ג' מספרים</ref> | ||
|- | |- | ||
|According to this procedure for every number. | |According to this procedure for every number. | ||
| − | |style="text-align:right;"|ועל זה הסדר | + | |style="text-align:right;"|ועל זה הסדר כל {{#annot:term|228|m9oU}}החשבון{{#annotend:m9oU}}‫<ref group=I>הסדר: P1051 הסדר החשבון<br> |
| + | זה הסדר: P1029 סדר זה<br> | ||
| + | החשבון: III חשבון</ref> | ||
|- | |- | ||
|See, whether the number consists of one or many [digits]. | |See, whether the number consists of one or many [digits]. | ||
| − | |style="text-align:right;"|וראה<ref group=I>וראה: V397 נראה< | + | |style="text-align:right;"|וראה אם המספר אחד או רבים‫<ref group=I>וראה: V397 נראה<br> |
| + | אחד: O187 האחד<br> | ||
| + | רבים: O187 הרבים; P1051 <sup>ה</sup>רבים</ref> | ||
|- | |- | ||
!<span style="color:green">Multiplication of evens and odds</span> | !<span style="color:green">Multiplication of evens and odds</span> | ||
| Line 577: | Line 1,260: | ||
| | | | ||
*If it is an even number, the product is also an even number. <math>\scriptstyle2a\times2b</math> | *If it is an even number, the product is also an even number. <math>\scriptstyle2a\times2b</math> | ||
| − | |style="text-align:right;"|אם<ref group=I>אם: O187; P1050 om.; P1029 ואם< | + | |style="text-align:right;"|אם הוא מספר זוג גם המחובר יהיה זוג‫<ref group=I>אם: O187; P1050 om.; P1029 ואם<br> |
| + | אם הוא: Mo30; III והוא<br> | ||
| + | זוג: V397 om.</ref> | ||
|- | |- | ||
| | | | ||
| Line 585: | Line 1,270: | ||
| | | | ||
:The essence of the even number is in the units. | :The essence of the even number is in the units. | ||
| − | |style="text-align:right;"|וטעם הזוג<ref group=I>הזוג: O187 הזוג והנפרד</ref> | + | |style="text-align:right;"|וטעם הזוג הוא באחדים‫<ref group=I>הזוג: O187 הזוג והנפרד</ref> |
|- | |- | ||
| | | | ||
| Line 593: | Line 1,278: | ||
| | | | ||
*If one number is even and the other is odd, meaning that it is not even, whichever one of them is, the product is also an even number. <math>\scriptstyle2a\times\left(2b-1\right)</math> | *If one number is even and the other is odd, meaning that it is not even, whichever one of them is, the product is also an even number. <math>\scriptstyle2a\times\left(2b-1\right)</math> | ||
| − | |style="text-align:right;"|ואם<ref group=I>ואם: V398 ואף אם< | + | |style="text-align:right;"|ואם המספר האחד זוג והשני נפרד והטעם שאינו זוג איזה מהם שיהיה גם המחובר יהיה זוג‫<ref group=I>ואם: V398 ואף אם<br> |
| + | האחד: V397 marg. אחד<br> | ||
| + | זוג: V398; P1050; III הוא זוג<br> | ||
| + | נפרד: O187 נפרד המחובר זוג; W152 הוא נפרד<br> | ||
| + | והטעם: III הטעם<br> | ||
| + | שאינו: O187 כשהוא<br> | ||
| + | והטעם שאינו זוג: P1050 om.<br> | ||
| + | מהם: P1029; V398 מהן</ref> | ||
|- | |- | ||
| | | | ||
*If the one number is odd and the other is also, the product is also odd. <math>\scriptstyle\left(2a-1\right)\times\left(2b-1\right)</math> | *If the one number is odd and the other is also, the product is also odd. <math>\scriptstyle\left(2a-1\right)\times\left(2b-1\right)</math> | ||
| − | |style="text-align:right;"|ואם המספר<ref group=I>המספר: Mo30 om.< | + | |style="text-align:right;"|ואם המספר האחד נפרד וגם כן האחר גם המחובר יהיה נפרד‫<ref group=I>המספר: Mo30 om.<br> |
| + | האחד: V397 אחד<br> | ||
| + | נפרד: P1051 יהיה נפרד<br> | ||
| + | וגם: V398; III גם<br> | ||
| + | כן: Lo27153 top<br> | ||
| + | וגם כן: O187 ג"כ<br> | ||
| + | האחר: P1029; P1051; V397 האחר נפרד<br> | ||
| + | גם: P1050 הנה גם כן; W152; W194 גם כן<br> | ||
| + | המחובר: Mo30 המרובע<br> | ||
| + | נפרד: Lo27153 זוג marg. ד"ת נפרד</ref> | ||
|- | |- | ||
| | | | ||
| Line 606: | Line 1,307: | ||
|- | |- | ||
|Know that if the numbers to be multiplied by each other are many, you have to multiply them by writing the 9 characters that I have showed you. | |Know that if the numbers to be multiplied by each other are many, you have to multiply them by writing the 9 characters that I have showed you. | ||
| − | |style="text-align:right;"|ודע כי אם היו<ref group=I>היו: Mo30 יהיו< | + | |style="text-align:right;"|ודע כי אם היו המספרים הנכפלים אלה על אלה רבים אתה צריך לכפול אותם במכתב ט' {{#annot:term|204|caYJ}}אותיות{{#annotend:caYJ}} שהראיתיך‫<ref group=I>היו: Mo30 יהיו<br> |
| + | המספרים: Mo30; III מספרים; P1051; V397 om.<br> | ||
| + | הנכפלים: V398; P1050; III om.<br> | ||
| + | אלה: V397; P1051 top האלה<br> | ||
| + | לכפול: V397 לכתוב<br> | ||
| + | לכפול אותם: P1029 לכלם<br> | ||
| + | במכתב: Mo30 במספר<br> | ||
| + | שהראיתיך: V397 marg.</ref> | ||
|- | |- | ||
| | | | ||
| Line 614: | Line 1,322: | ||
| | | | ||
:The paved way is that you put the smaller number in the top row; the meaning of the smaller number is the one whose decade is smaller; do not consider the units. | :The paved way is that you put the smaller number in the top row; the meaning of the smaller number is the one whose decade is smaller; do not consider the units. | ||
| − | |style="text-align:right;"|והדרך הסלולה<ref group=I>הסלולה: P1050 הצל הסלולה; V398 הצלולה< | + | |style="text-align:right;"|והדרך הסלולה שתשים טורי המספר המעט עליונים ופירוש המעט בחשבון הכלל ולא תחוש מן הפרטים‫<ref group=I>הסלולה: P1050 הצל הסלולה; V398 הצלולה<br> |
| + | המעט: Mo30 marg.<br> | ||
| + | עליונים: P1050; P1051; V398; III העליונים<br> | ||
| + | ופירוש: O187 פי‫'<br> | ||
| + | המעט: P1050 המעט marg. בהם<br> | ||
| + | ולא: Lo27153 לא; Mo30 ואל<br> | ||
| + | מן: P1051 om.</ref> | ||
|- | |- | ||
| | | | ||
| Line 630: | Line 1,344: | ||
| | | | ||
:If, however, the number, whose decade is smaller, consists of more digits than the number whose decade is greater, then put this in the top row without consideration. | :If, however, the number, whose decade is smaller, consists of more digits than the number whose decade is greater, then put this in the top row without consideration. | ||
| − | |style="text-align:right;"|ואם<ref group=I>ואם: P1050 ואף <sup>אם</sup>< | + | |style="text-align:right;"|ואם החשבון שכללו קטן הם יותר מספרים מחשבון שכללו גדול שים אותם עליונים ולא תחוש‫<ref group=I>ואם: P1050 ואף <sup>אם</sup><br> |
| + | קטן: P1051 קטון<br> | ||
| + | הם: Lo27153 הוא<br> | ||
| + | גדול: P1051 marg. הגדול<br> | ||
| + | אותם: P1029 אותה</ref> | ||
|- | |- | ||
|If you would do the other way around, it does no harm, just confuses the student a little. | |If you would do the other way around, it does no harm, just confuses the student a little. | ||
| − | |style="text-align:right;"|ואלו<ref group=I>ואלו: Mo30; P1050 ואם< | + | |style="text-align:right;"|ואלו היית עושה להפך לא יזיק רק יתבלבל מעט על התלמיד‫<ref group=I>ואלו: Mo30; P1050 ואם<br> |
| + | היית: Mo30; P1029; P1051 היתה<br> | ||
| + | ואלו היית: P1029 ואלו היתה ואלו היתה<br> | ||
| + | יזיק: O187 היה מזיק<br> | ||
| + | מעט: O187 ממנו<br> | ||
| + | התלמיד: P1029 התלמידים<br> | ||
| + | על התלמיד: Mo30 המלמד</ref> | ||
|- | |- | ||
| | | | ||
*After you put the digits, as many as they are, in the top row and the others in the bottom row, multiply the first from the top row by the first from the bottom row, and write the result corresponding to the first [that in the] top row. | *After you put the digits, as many as they are, in the top row and the others in the bottom row, multiply the first from the top row by the first from the bottom row, and write the result corresponding to the first [that in the] top row. | ||
| − | |style="text-align:right;"|ואחר שתשים המספרים כמה שיהיו בטור העליון<ref group=I>העליון: P1051 <sup>ה</sup>עליון< | + | |style="text-align:right;"|ואחר שתשים המספרים כמה שיהיו בטור העליון והאחרים בטור השפל כפול הראשון של הטור העליון על הראשון שבטור השפל והעולה כתוב אותו כנגד הטור הראשון העליון‫<ref group=I>העליון: P1051 <sup>ה</sup>עליון<br> |
| + | והאחרים: O187 והא<sup>ח</sup>דים<br> | ||
| + | הראשון: P1029 <s>העליון</s> הראשון<br> | ||
| + | של: P1050; III מן<br> | ||
| + | הטור: Mo30; O187; P1051 טור<br> | ||
| + | הראשון: P1029 ראשון<br> | ||
| + | שבטור: O187; P1051; V397; III בטור; P1050 <sup>ש</sup>בטור<br> | ||
| + | השפל: W152 <s>השפל</s> <sup>השפל</sup>; P1029 top<br> | ||
| + | והעולה כתוב: Mo30 והעולה כתוב marg. וכתוב העולה<br> | ||
| + | כפול הראשון ...והעולה כתוב: Mo30 marg.<br> | ||
| + | הטור: O187 המספר<br> | ||
| + | הראשון: Mo30; P1051; V397 om.<br> | ||
| + | העליון: W152 העליון הראשון; P1050 העליון marg. בטור השלישי</ref> | ||
|- | |- | ||
| | | | ||
:Then, multiply the first top digit by the second bottom digit and write [the result] in the third row corresponding to the second top digit. | :Then, multiply the first top digit by the second bottom digit and write [the result] in the third row corresponding to the second top digit. | ||
| − | |style="text-align:right;"|ואחר כן<ref group=I>ואחר כך: Mo30 אח"כ< | + | |style="text-align:right;"|ואחר כן כפול המספר הראשון העליון על המספר השני השפל וכתוב בטור השלישי כנגד המספר השני העליון‫<ref group=I>ואחר כך: Mo30 אח"כ<br> |
| + | המספר: P1029 מספר<br> | ||
| + | הראשון: Mo30 om.<br> | ||
| + | המספר: P1029 מספר<br> | ||
| + | השלישי: O187; P1029 שלישי<br> | ||
| + | המספר: Mo30 מספר; P1051 twice</ref> | ||
|- | |- | ||
| | | | ||
| Line 653: | Line 1,394: | ||
| | | | ||
::If, when you multiply the first upper digit by its corresponding one in the bottom [row] there is a decade and units in the product, write the units in the place to which it belongs and write the number of the decade as the following digit. | ::If, when you multiply the first upper digit by its corresponding one in the bottom [row] there is a decade and units in the product, write the units in the place to which it belongs and write the number of the decade as the following digit. | ||
| − | |style="text-align:right;"|ואם כאשר תכפול הראשון העליון<ref group=I>העליון: O187 של העליון; III om.; P1050 top< | + | |style="text-align:right;"|ואם כאשר תכפול הראשון העליון על שכנגדו בשפל ויתחבר במספר כלל ופרט תכתוב הפרט במקום הראוי לו והכלל במספרו תכתבנו בחשבון שהוא אחריו‫<ref group=I>העליון: O187 של העליון; III om.; P1050 top<br> |
| + | ויתחבר: V397 ויתחייב<br> | ||
| + | והכלל: P1029 והכל<br> | ||
| + | במספרו: V397 במספר</ref> | ||
|- | |- | ||
| | | | ||
*After you have finished multiplying the first digit of the top row by all the digits of the bottom row, start to multiply the second digit of the top row by the first digit of the bottom row and write the result in the third row corresponding to the second upper [digit]. | *After you have finished multiplying the first digit of the top row by all the digits of the bottom row, start to multiply the second digit of the top row by the first digit of the bottom row and write the result in the third row corresponding to the second upper [digit]. | ||
| − | |style="text-align:right;"|ואחר שתשלים<ref group=I>שתשלים: P1029 שתשים< | + | |style="text-align:right;"|ואחר שתשלים לכפול החשבון הראשון של הטור העליון על כל מספרי הטור השפל תחל לכפול המספר השני של הטור העליון על מספר הראשון של הטור השפל והעולה כתבהו בטור השלישי כנגד השני העליון‫<ref group=I>שתשלים: P1029 שתשים<br> |
| + | הטור: Lo27153; Mo30; O187; P1050; V398 טור<br> | ||
| + | מספרי: P1051 מספר<br> | ||
| + | הטור: Mo30 om.<br> | ||
| + | לכפול: Lo27153 <s>לשמור</s> לכפול<br> | ||
| + | המספר: P1029 <s>המספר</s> המספר<br> | ||
| + | הטור: Mo30 טור<br> | ||
| + | של הטור: V397 מטור<br> | ||
| + | מספר: O187; P1051 המספר<br> | ||
| + | הטור: Mo30 טור; V397; P1051; V398 מספר<br> | ||
| + | של הטור: O187 מטור; P1029 om.<br> | ||
| + | כתבהו: P1050 כתבנו; P1051; V397 תכתבנו</ref> | ||
|- | |- | ||
| | | | ||
:Then, multiply the second [of the] top by the second that is in the bottom row and write it in the third row as the third digit, which is second to the digit from with which you have now started. | :Then, multiply the second [of the] top by the second that is in the bottom row and write it in the third row as the third digit, which is second to the digit from with which you have now started. | ||
| − | |style="text-align:right;"|ואחר<ref group= | + | |style="text-align:right;"|ואחר כן תכפול השני העליון על שני שבטור השפל ותכתבהו בטור השלישי במספר השלישי<ref group=note>במספר השלישי: V398 marg. פי' במעלה השלי'</ref> שהוא שני למספר שהחלות עתה ממנו‫<ref group=I>ואחר: P1051 ואחרי<br> |
| + | השני: P1050 השני marg. שבטור<br> | ||
| + | שני: P1050 השני<br> | ||
| + | ותכתבהו: O187 והעולה תכתבהו; P1050 וכתבנו<br> | ||
| + | כנגד השני ... בטור השלישי: P1029 om.<br> | ||
| + | במספר השלישי: P1051; V397 om.<br> | ||
| + | שני: P1051 שלישי<br> | ||
| + | למספר: O187 של מספר<br> | ||
| + | שהחלות: V397 שהתחלנו<br> | ||
| + | ממנו: O187 ממנו וכן עד השלימך מספרי הטור השפל</ref> | ||
|- | |- | ||
| | | | ||
*Then, start with the third upper digit, multiply it by the first of the bottom row, and write the result corresponding to the third [digit] with which you have started. | *Then, start with the third upper digit, multiply it by the first of the bottom row, and write the result corresponding to the third [digit] with which you have started. | ||
| − | |style="text-align:right;"|ואחר<ref group=I>ואחר: P1051 ואחרי< | + | |style="text-align:right;"|ואחר כן תחל במספר השלישי העליון לכפול אותו על הראשון שבטור השפל והעולה תכתבהו כנגד טור השלישי שהחילות ממנו‫<ref group=I>ואחר: P1051 ואחרי<br> |
| + | העליון: Mo30 בטור העליון<br> | ||
| + | לכפול אותו: P1029 לכפלו<br> | ||
| + | תכתבהו: V397; V398 תכתבנו<br> | ||
| + | טור: Mo30 הטור; O187; P1051 om.<br> | ||
| + | כנגד טור: Lo27153 בטור<br> | ||
| + | שהחלות: Lo27153 שהחלו<s>ת</s><sup>ת</sup></ref> | ||
|- | |- | ||
|This is the procedure for all endlessly with the rule that the units are into the lower rank and the decade following it in the next rank. | |This is the procedure for all endlessly with the rule that the units are into the lower rank and the decade following it in the next rank. | ||
| − | |style="text-align:right;"|וככה<ref group=I>ממנו וככה: V397 וככה ממנו< | + | |style="text-align:right;"|וככה המשפט לכלם עד אין קץ עם משפט הפרט להיות התחתון והכלל שיבא אחריו בטור השני לו‫<ref group=I>ממנו וככה: V397 וככה ממנו<br> |
| + | המשפט: Mo30; O187 משפט<br> | ||
| + | לכלם: O187 כולם<br> | ||
| + | עד: Mo30 כי<br> | ||
| + | משפט: Mo30; P1029; P1050; P1051; III משפטי; V397 משפטי משפטי<br> | ||
| + | הפרט: Lo27153 <s>הפרט</s> הפרט; P1051 פרט<br> | ||
| + | התחתון: O187 תחתיו; P1029; P1050; P1051; V397; III תחתון<br> | ||
| + | והכלל: P1051; V397 עם הכלל<br> | ||
| + | שהחילות ... והכלל: V398 om.<br> | ||
| + | שיבא: Mo30 הבא; V398 ושיבא; W152 כשיבא<br> | ||
| + | בטור: O187 במספר</ref> | ||
|- | |- | ||
|If there is zero, whether in the top row or in the bottom row, the rule is to write it in its appropriate position, as is the rule for all numbers next to it. | |If there is zero, whether in the top row or in the bottom row, the rule is to write it in its appropriate position, as is the rule for all numbers next to it. | ||
| − | |style="text-align:right;"|ואם היה<ref group= | + | |style="text-align:right;"|ואם היה גלגל בין בטור העליון בין בטור השפל משפטו לכתבו במקום הראוי לו<ref group=note>V398 marg. פי' להכיר מה שבא אחריו מן המספר באיזה מעלה היא</ref> כמשפט כל המספרים שעליו‫<ref group=I>היה: O187 יהיה<br> |
| + | בטור: Mo30 הטור; V397 <sup>ב</sup>טור<br> | ||
| + | בין: Mo30; P1051; V397; V398 ובין<br> | ||
| + | בטור: Mo30 הטור; V397 <sup>ב</sup>טור<br> | ||
| + | משפטו: Lo27153; P1050 משפט<br> | ||
| + | במקום: Mo30 בטור<br> | ||
| + | שעליו: P1029 שעליו ואחריו; W152 שלעליו marg. שעליו</ref> | ||
|- | |- | ||
| | | | ||
*Then, start adding up the interim products of the top row with the bottom: | *Then, start adding up the interim products of the top row with the bottom: | ||
| − | |style="text-align:right;"|ואחר כן תחל<ref group= | + | |style="text-align:right;"|ואחר כן תחל לחבר מה שעלה בטור העליון עם השפל‫<ref group=note>P1051 marg. פי'ק' ר"ל מכפילת הטור העליון עם השפל</ref>‫<ref group=I>תחל: Mo30 תשוב<br> |
| + | לחבר: Lo27153 <s>לחסר</s> לחבר<br> | ||
| + | שעלה: P1051; V397 שעולה<br> | ||
| + | בטור: O187 מטור</ref> | ||
|- | |- | ||
| | | | ||
:*If there are no tens there, write down the sum. | :*If there are no tens there, write down the sum. | ||
| − | |style="text-align:right;"|אם<ref group=I>אם: Mo30 כי; O187 ואם< | + | |style="text-align:right;"|אם אין בו עשרות תכתוב מה שהוא בחבור‫<ref group=I>אם: Mo30 כי; O187 ואם<br> |
| + | בו: O187 לו; V397 om.<br> | ||
| + | עשרות: Mo30 עשיריות<br> | ||
| + | שהוא בחבור: O187 שבחבור</ref> | ||
|- | |- | ||
| | | | ||
| Line 687: | Line 1,479: | ||
| | | | ||
:*If there is more, write the excess aside from the sum you have and instead of the ten write one next to it. | :*If there is more, write the excess aside from the sum you have and instead of the ten write one next to it. | ||
| − | |style="text-align:right;"|ואם יש בו<ref group=I>בו: O187; V397 לו< | + | |style="text-align:right;"|ואם יש בו יותר כתוב היותר מבחוץ בחבור שיש לך ובמקום העשרה כתוב אחד שני לו נוסף‫<ref group=I>בו: O187; V397 לו<br> |
| + | כתוב: P1029 om.<br> | ||
| + | היותר: O187; V397 יותר; P1050 האחד<br> | ||
| + | מבחוץ: Mo30 מחוץ<br> | ||
| + | בחבור: P1029 om.<br> | ||
| + | מבחוץ בחבור שיש לך: V398 בחבור שיש לך מבחוץ<br> | ||
| + | שני: Lo27153 שיש; Mo30 marg.<br> | ||
| + | לו: Lo27153 לו marg. לך; P1051 top</ref> | ||
|- | |- | ||
| | | | ||
:Proceed so with all the interim products of the top and bottom rows and take out the excesses aside from the tens. | :Proceed so with all the interim products of the top and bottom rows and take out the excesses aside from the tens. | ||
| − | |style="text-align:right;"|וכן תעשה<ref group=I>תעשה: Mo30 עשרה< | + | |style="text-align:right;"|וכן תעשה לכל היוצאים מהטור העליון והשפל והוצא הנותר מעשרות מבחוץ‫<ref group=I>תעשה: Mo30 עשרה<br> |
| + | היוצאים: O187 היוצא<br> | ||
| + | מהטור: Mo30; P1029 מן הטור; O187 מהטורים; P1051 מן הטורים<br> | ||
| + | העליון: P1051 העליונים<br> | ||
| + | והשפל: Mo30; P1050; V398 והטור השפל<br> | ||
| + | והוצא: P1029 והיוצא<br> | ||
| + | הנותר: V398 הנזכר marg. הנותר<br> | ||
| + | מעשרות: O187; P1029; V398; III מהעשרות</ref> | ||
|- | |- | ||
| | | | ||
*After you know how much the sum in the third row is, count its ranks and see: if they are as much as the number of the ranks of the two upper rows minus one, know that your calculation is correct. | *After you know how much the sum in the third row is, count its ranks and see: if they are as much as the number of the ranks of the two upper rows minus one, know that your calculation is correct. | ||
| − | |style="text-align:right;"|ואחר שידעת כמה הוא | + | |style="text-align:right;"|ואחר שידעת כמה הוא המחובר בטור השלישי ספור מעלותיו וראה אם היו כמספר מעלות השנים טורים העליונים ממנו בחסרון אחד תדע כי חשבונך אמת‫<ref group=note>V398 marg. בספר אחר ראיתי הדרך אחרת</ref>‫<ref group=I>הוא: P1029 om.<br> |
| + | היו: P1029 הם<br> | ||
| + | השנים: P1029 השני<br> | ||
| + | העליונים: V398 <s>עול</s> עליונים; O187; P1029; V397; III עליונים<br> | ||
| + | אחד: Mo30 האחד<br> | ||
| + | תדע: O187; W152 דע</ref> | ||
|- | |- | ||
| | | | ||
:If the product of last digit in the top row multiplied by the last digit in the bottom row is a decade, the number of ranks in the third row is the same as to the number of [ranks of] the two upper rows without the subtraction of one. | :If the product of last digit in the top row multiplied by the last digit in the bottom row is a decade, the number of ranks in the third row is the same as to the number of [ranks of] the two upper rows without the subtraction of one. | ||
| − | |style="text-align:right;"|ואם המספר<ref group=I>המספר: Mo30; P1029; P1050; P1051; V398 היה המספר; O187 יהיה מספר; V397 יהיה המספר< | + | |style="text-align:right;"|ואם המספר האחרון בטור העליון הנכפל במספר האחרון בטור השפל ממנו יוצא אל כלל<ref group=note>יוצא אל כלל: Mo30 marg. ר"ל מגיע עד מדרגת העשרות</ref> יהיה מספר מעלות הטור השלישי כמספר שני טורים העליונים בלי מגרעת אחד‫<ref group=I>המספר: Mo30; P1029; P1050; P1051; V398 היה המספר; O187 יהיה מספר; V397 יהיה המספר<br> |
| + | האחרון: O187 אחרון<br> | ||
| + | האחרון: Mo30 העליון; O187 אחרון<br> | ||
| + | ממנו: V397 <s>ממנו</s> ממנו<br> | ||
| + | כלל: P1050; III הכלל<br> | ||
| + | יהיה: P1029 היה<br> | ||
| + | מספר: P1029 המספר<br> | ||
| + | הטור: V397 om.<br> | ||
| + | מעלות הטור: Mo30 המעלות טור; P1051 המעלות בטור<br> | ||
| + | הטור השלישי: P1029 טור ג‫'<br> | ||
| + | טורים: P1050; P1051; V398 הטורים<br> | ||
| + | העליונים: P1029; V397 עליונים<br> | ||
| + | בלי: III בלתי<br> | ||
| + | אחד: P1029; P1050 אחת</ref> | ||
|- | |- | ||
!<span style="color:green">Check: casting out by 9</span> | !<span style="color:green">Check: casting out by 9</span> | ||
| Line 705: | Line 1,529: | ||
|- | |- | ||
|Finally, check it through the scales. | |Finally, check it through the scales. | ||
| − | |style="text-align:right;"|‫<ref group=I>P1050; III example before the check< | + | |style="text-align:right;"|ובחן באחרונה במאזנים‫<ref group=I>P1050; III example before the check<br> |
| + | ובחן: O187; P1051; V397 ובחון<br> | ||
| + | במאזנים: P1029; P1051 מאזנים</ref> | ||
|- | |- | ||
|You proceed as follows: | |You proceed as follows: | ||
| − | |style="text-align:right;"|וככה<ref group=I>וככה: Mo30 וכה; V398; P1050; III ככה< | + | |style="text-align:right;"|וככה תעשה‫<ref group=I>וככה: Mo30 וכה; V398; P1050; III ככה<br> |
| + | תעשה: V398; P1050; III om.</ref> | ||
|- | |- | ||
|Consider every digit in the top row in any rank as if it were units, sum them and cast out the nines from the sum if it is more than 9, or less than it write alone, and this is the scale of the top row. | |Consider every digit in the top row in any rank as if it were units, sum them and cast out the nines from the sum if it is more than 9, or less than it write alone, and this is the scale of the top row. | ||
| − | |style="text-align:right;"|חשוב<ref group= | + | |style="text-align:right;"|<div id="as_if_they_are_units"></div>חשוב כל חשבון שתמצא בטור העליון באיזו מעלה שיהיה כאילו הם אחדים‫<ref group=note>[[Excerpts_Attributed_to_Immanuel_Bonfils#As_if_they_are_units|<span style=color:blue>commentary: as if they are units</span>]]</ref> וחברם והוצא המחובר ט' ט' אם יותר ט' או פחות ממנו כתוב אותו לבדו והוא מאזני הטור העליון‫<ref group=I>חשוב: O187; V397 הוצא<br> |
| + | חשבון: P1029; P1051; V398 החשבון<br> | ||
| + | באיזו: Mo30; O187; P1051; V397 באיזה<br> | ||
| + | שיהיה: W152 שיהיו<br> | ||
| + | המחובר: P1051 marg.; P1050 המספר<br> | ||
| + | אם: Mo30; V398 ואם<br> | ||
| + | יותר: Mo30 ישאר; P1029 יוסר; P1050 הוא יותר אחד<br> | ||
| + | ט‫': P1050; W152 מט‫'<br> | ||
| + | או: Lo27153 <s>או</s> או<br> | ||
| + | לבדו: O187 לבד; V397 לבדד<br> | ||
| + | והוא: V397 והם</ref> | ||
|- | |- | ||
|Do the same with the scale of the bottom row until you know how much its scale is. | |Do the same with the scale of the bottom row until you know how much its scale is. | ||
| − | |style="text-align:right;"|ככה<ref group=I>ככה: Mo30 וכך; V398; P1050; III וכן< | + | |style="text-align:right;"|ככה תעשה למאזני הטור השפל עד שתדע כמה המאזנים שלו‫<ref group=I>ככה: Mo30 וכך; V398; P1050; III וכן<br> |
| + | למאזני הטור: P1051 לטור מאזני<br> | ||
| + | המאזנים: O187 מאזנים<br> | ||
| + | שלו: W152 שלא</ref> | ||
|- | |- | ||
|Multiply the scale of the top row by the scale of the other row, then cast out the nines from the product and keep the remainder with you. | |Multiply the scale of the top row by the scale of the other row, then cast out the nines from the product and keep the remainder with you. | ||
| − | |style="text-align:right;"|וכפול מאזני הטור העליון<ref group=I>העליון: Mo30 השני< | + | |style="text-align:right;"|וכפול מאזני הטור העליון על מאזני הטור השני והנכפל הוציאהו ט' ט' והנשאר יהיה עמך שמור‫<ref group=I>העליון: Mo30 השני<br> |
| + | השני: P1050; III השפל<br> | ||
| + | על מאזני הטור השני: Mo30 om.<br> | ||
| + | והנכפל: P1029; V397 והכפל<br> | ||
| + | הוציאהו: Mo30 תוציאנו; O187; P1029; P1051; V397 הוציאנו; V398 הוציאו; W152 הוציאוהו<br> | ||
| + | והנשאר: Mo30 om.<br> | ||
| + | יהיה: Mo30 ויהיה; O187 שיהיה<br> | ||
| + | עמך: O187; V397 בידך</ref> | ||
|- | |- | ||
|If the scale of one of the rows is 9, do not weary yourself to find the scale of the other row, because it will always be casted out by 9. | |If the scale of one of the rows is 9, do not weary yourself to find the scale of the other row, because it will always be casted out by 9. | ||
| − | |style="text-align:right;"|ואם מאזני אחד מהטורים | + | |style="text-align:right;"|ואם מאזני אחד מהטורים יהיה ט' אל תיגע עצמך לבקש מאזני הטור האחר כי ט' יצא לעולם‫<ref group=I>מהטורים: III מן הטורים<br> |
| + | לעולם: V398 om.</ref> | ||
|- | |- | ||
|Then, examine the scale of the third row and see: if it is the same as the reserved, then your calculation is true, but if not, you were wrong. | |Then, examine the scale of the third row and see: if it is the same as the reserved, then your calculation is true, but if not, you were wrong. | ||
| − | |style="text-align:right;"|ואחר<ref group=I>ואחר: O187 ואחר כך; V397 ואחר כן; P1050 ואח"כ< | + | |style="text-align:right;"|ואחר בדוק מאזני הטור השלישי וראה אם היה שוה לשמור חשבונך אמת ואם לאו הנה טעית‫<ref group=I>ואחר: O187 ואחר כך; V397 ואחר כן; P1050 ואח"כ<br> |
| + | מאזני: W152 במאזני<br> | ||
| + | אם: Lo27153 כי אם<br> | ||
| + | שוה: Mo30; P1051 חשבונך שוה<br> | ||
| + | חשבונך: Mo30 אז תדע כי חשבונך; O187; V397 תדע כי חשבונך; P1029 יהיה חשבונך<br> | ||
| + | לאו: P1029; P1051 לא<br> | ||
| + | הנה: P1029 om.<br> | ||
| + | הנה טעית: Lo27153 <sup>הנה</sup> הוא טעות<br> | ||
| + | ואם לאו הנה טעית: O187; V397 om.</ref> | ||
|- | |- | ||
| | | | ||
*{{#annot:127×355|156|cmWh}}Example: we wish to multiply 127 by 355. | *{{#annot:127×355|156|cmWh}}Example: we wish to multiply 127 by 355. | ||
:<math>\scriptstyle127\times355</math> | :<math>\scriptstyle127\times355</math> | ||
| − | |style="text-align:right;"|‫<ref group=I>O187; P1051; V397; V398 example om.< | + | |style="text-align:right;"|<big>דמיון</big> זה רצינו לכפול קכ"ז על שנ"ה{{#annotend:cmWh}}‫<ref group=I>O187; P1051; V397; V398 example om.<br> |
| + | זה: Mo30; P1029; P1050 om.</ref> | ||
|- | |- | ||
| | | | ||
:We write 127 in the top row and the number 355 beneath it, each characters in its place, like this. | :We write 127 in the top row and the number 355 beneath it, each characters in its place, like this. | ||
| − | |style="text-align:right;"|וכתבנו<ref group=I>וכתבנו: Mo30 כתוב; P1050; III כתבנו; P1029 וכתו'< | + | |style="text-align:right;"|וכתבנו קכ"ז בטור העליון כזה ומספר שנ"ה תחתיו אות אות במקומו כזה‫<ref group=I>וכתבנו: Mo30 כתוב; P1050; III כתבנו; P1029 וכתו‫'<br> |
| + | קכ"ז בטור העליון: Mo30; P1029 בטור העליון קכ"ז<br> | ||
| + | כזה: Lo27153; Mo30; P1029; P1050 om.<br> | ||
| + | ומספר: P1029 ובשני<br> | ||
| + | אות אות: III כל אות ואות; W194 <sup>כל</sup> אות <sup>ואות</sup><br> | ||
| + | תחתיו אות אות במקומו: P1029 om.<br> | ||
| + | כזה: P1029 בזו הצורה; P1050; W152; W194 om.<br> | ||
| + | ומספר ...כזה: Mo30 ובשניה שנ"ה</ref> | ||
|- | |- | ||
| | | | ||
| Line 814: | Line 1,678: | ||
| | | | ||
:*We multiply 7 by 5; the result is 35. We write 5 in the first rank and 3, which is 30, in the second rank. | :*We multiply 7 by 5; the result is 35. We write 5 in the first rank and 3, which is 30, in the second rank. | ||
| − | |style="text-align:right;"|כפלנו<ref group=I>כפלנו: Mo30; P1029 נכפול< | + | |style="text-align:right;"|כפלנו ז' על ה' עלו ל"ה כתבנו ה' במעלה הראשונה וג' שהוא ל' במעלה השניה‫<ref group=I>כפלנו: Mo30; P1029 נכפול<br> |
| + | עלו: Mo30; P1029 ויהיו<br> | ||
| + | כתבנו: Mo30 כתוב; P1029 תכתוב<br> | ||
| + | שהוא ל‫': Mo30; P1029 om.<br> | ||
| + | השניה: P1050; III השנית<br> | ||
| + | במעלה השניה: Mo30; P1029 בשנית</ref> | ||
|- | |- | ||
| | | | ||
:*We also multiply 7 by the second bottom 5; the result is 35. We write 5 in the second rank beneath the 3 and 3 in the third [rank]. | :*We also multiply 7 by the second bottom 5; the result is 35. We write 5 in the second rank beneath the 3 and 3 in the third [rank]. | ||
| − | |style="text-align:right;"|עוד כפלנו<ref group=I>כפלנו: Mo30; P1029 תכפול; III נכפול< | + | |style="text-align:right;"|עוד כפלנו ז' על ה' השני התחתון עלו ל"ה כתבנו ה' במעלה השנית תחת ג' וג' בשלישית‫<ref group=I>כפלנו: Mo30; P1029 תכפול; III נכפול<br> |
| + | השני התחתון: Mo30 פעם אחרת; P1029 שלו האחרת<br> | ||
| + | עלו: P1029 יעלו<br> | ||
| + | עלו ל"ה: P1050; III om.<br> | ||
| + | כתבנו: Mo30; P1029 כתוב; P1050; III וכתבנו<br> | ||
| + | ה‫': Mo30; P1029 הה‫'<br> | ||
| + | השנית: P1050 שנית<br> | ||
| + | ג‫': Mo30; P1029 הג‫'<br> | ||
| + | תחת ג‫': P1050; III om.<br> | ||
| + | בשלישית: P1050 במעלה שלישית; III במעלה השלישית</ref> | ||
|- | |- | ||
| | | | ||
:*We also multiply the first 7 by the bottom 3; the result is 21. We write 1 in the third [rank] beneath 3 and 2 in the fourth [rank]. | :*We also multiply the first 7 by the bottom 3; the result is 21. We write 1 in the third [rank] beneath 3 and 2 in the fourth [rank]. | ||
| − | |style="text-align:right;"|עוד כפלנו<ref group=I>כפלנו: Mo30; P1029 תכפול< | + | |style="text-align:right;"|עוד כפלנו ז' הראשון על ג' התחתון עלו כ"א כתבנו א' בשלישית תחת ג' וב' ברביעית‫<ref group=I>כפלנו: Mo30; P1029 תכפול<br> |
| + | הראשון: P1050; III om.<br> | ||
| + | התחתון: III התחתון השלישית; P1050 התחתון השלישי<br> | ||
| + | עלו: P1029 יעלו<br> | ||
| + | כתבנו: Mo30; P1029 כתוב<br> | ||
| + | א‫': P1029 הא‫'; P1050 <s>ב</s> א‫'<br> | ||
| + | תחת ג‫': P1029 הג‫'; P1050; III om.<br> | ||
| + | ברביעית: P1029 <s>רב</s> ברביעית</ref> | ||
|- | |- | ||
| colspan=2| | | colspan=2| | ||
| Line 841: | Line 1,726: | ||
| | | | ||
:*We also multiply the middle upper 2 by the first 5 from the bottom [row]; the result is 10. We write 1 in the third ]rank] beneath the 1. | :*We also multiply the middle upper 2 by the first 5 from the bottom [row]; the result is 10. We write 1 in the third ]rank] beneath the 1. | ||
| − | |style="text-align:right;"|עוד<ref group=I>עוד: W152 ועוד< | + | |style="text-align:right;"|עוד כפלנו ב' האמצעי העליון על ה' הראשון מן התחתון עלו י' כתבנו א' בשלישית תחת הא‫'‫<ref group=I>עוד: W152 ועוד<br> |
| + | כפלנו: Mo30 תכפול; III נכפול; P1029 כפול<br> | ||
| + | העליון: P1029 מהטור העליון<br> | ||
| + | הראשון: W152; W194 om.<br> | ||
| + | ה' הראשון: Mo30 הראשנה; P1029 הא‫'<br> | ||
| + | מן: III om.; P1050 top<br> | ||
| + | התחתון: Mo30 הטור התחתון<br> | ||
| + | עלו: P1029 ועלו<br> | ||
| + | כתבנו: Mo30; P1029 כתוב<br> | ||
| + | כתבנו א' בשלישית: III והיה ראוי לכתבו<br> | ||
| + | א‫': Mo30 om.</ref> | ||
|- | |- | ||
| | | | ||
:*We also multiply the upper 2 by the second lower 5; the result is also 10. We write 1 beneath 2 in the fourth [rank]. | :*We also multiply the upper 2 by the second lower 5; the result is also 10. We write 1 beneath 2 in the fourth [rank]. | ||
| − | |style="text-align:right;"|עוד כפלנו<ref group=I>כפלנו: Mo30; P1029 כפול; P1050; III נכפול< | + | |style="text-align:right;"|עוד כפלנו ב' העליון על ה' השנית התחתון היו גם כן י' כתבנו א' תחת ב' ברביעית‫<ref group=I>כפלנו: Mo30; P1029 כפול; P1050; III נכפול<br> |
| + | ב‫': Mo30; P1029 הב‫'<br> | ||
| + | העליון: Mo30 האמצעי; P1029 בעצמה<br> | ||
| + | ה‫': P1029 הה‫'<br> | ||
| + | ה' הראשון ... ב' העליון על ה‫': P1029 marg.<br> | ||
| + | השנית: P1029 האמצעי; P1050 השני<br> | ||
| + | ה' השנית: Mo30 הה' השניים<br> | ||
| + | התחתון: Mo30 של מטה; P1029 מהטור התחתון<br> | ||
| + | היו: P1029 יעלו; P1050; III יהיו<br> | ||
| + | גם כן: Mo30; P1029 om.<br> | ||
| + | כתבנו: P1029 כתוב; P1050; III נכתוב<br> | ||
| + | א‫': P1029 הא‫'<br> | ||
| + | כתבנו א‫': Mo30 ושים אותם; W194 אלה<br> | ||
| + | תחת ב': P1050; III om.<br> | ||
| + | ברביעית: Mo30 om.<br> | ||
| + | תחת ב' ברביעית: P1029 ברביעית תחת ב‫'</ref> | ||
|- | |- | ||
| | | | ||
:*We also multiply the top 2 by the bottom 3; the result is 6. We write it under 1 in the fourth [rank]. | :*We also multiply the top 2 by the bottom 3; the result is 6. We write it under 1 in the fourth [rank]. | ||
| − | |style="text-align:right;"|עוד כפלנו<ref group=I>כפלנו: Mo30; P1029 כפול; P1050; III נכפול< | + | |style="text-align:right;"|עוד כפלנו ב' העליון על ג' התחתון והיו ו' כתבנו אותו תחת א' ברביעית‫<ref group=I>כפלנו: Mo30; P1029 כפול; P1050; III נכפול<br> |
| + | ב‫': Mo30 הב‫'<br> | ||
| + | העליון: Mo30 האמצעי; P1029 האמצעי מהטור העליון<br> | ||
| + | ג‫': Mo30 הג‫'<br> | ||
| + | התחתון: Mo30; P1050 om.; P1029 התחתון מן הטור השפל<br> | ||
| + | והיו: Mo30 ויהיו; P1029 יעלו; P1050; III עלו<br> | ||
| + | כתבנו אותו: Mo30 ושים אותם; P1029 כתבהו; P1050; III ונכתבנו<br> | ||
| + | א‫': Lo27153 <s>ה</s>א'<br> | ||
| + | תחת א‫': Mo30 om.<br> | ||
| + | ברביעית: Mo30 במדרגה <s>חמישית</s> <sup>ד‫'</sup><br> | ||
| + | תחת א' ברביעית: P1029 ברביעית תחת הב‫'<br> | ||
| + | עוד כפלנו ב' העליון ... ברביעית: Mo30 marg.</ref> | ||
|- | |- | ||
| colspan=2| | | colspan=2| | ||
| Line 870: | Line 1,791: | ||
| | | | ||
:*We also multiply the last upper 1 by the first lower 5; the result is 5. We write it in the third [rank]. | :*We also multiply the last upper 1 by the first lower 5; the result is 5. We write it in the third [rank]. | ||
| − | |style="text-align:right;"|עוד כפלנו<ref group=I>כפלנו: Mo30; P1029 תכפול; P1050; III נכפול< | + | |style="text-align:right;"|עוד כפלנו א' העליון האחרון על ה' הראשון התחתון עלו ה' כתבנוהו בשלישית‫<ref group=I>כפלנו: Mo30; P1029 תכפול; P1050; III נכפול<br> |
| + | האחרון: P1050 om.<br> | ||
| + | העליון האחרון: Lo27153 האחרון העליון<br> | ||
| + | ה‫': P1029 הה‫'<br> | ||
| + | התחתון: Mo30; P1029 מטור התחתון<br> | ||
| + | הראשון התחתון: P1050; III התחתון הראשון<br> | ||
| + | עלו: P1029 יעלו<br> | ||
| + | עלו ה‫': Mo30 om.<br> | ||
| + | כתבנוהו: Mo30; P1029 כתבהו; P1050 כתבנו<br> | ||
| + | בשלישית: Mo30 בג‫'</ref> | ||
|- | |- | ||
| | | | ||
:*We also multiply 1 by the second lower 5; the result is 5. We write it in the fourth [rank]. | :*We also multiply 1 by the second lower 5; the result is 5. We write it in the fourth [rank]. | ||
| − | |style="text-align:right;"|עוד כפלנו<ref group=I>כפלנו: Mo30; P1029 כפול; P1050; III נכפול< | + | |style="text-align:right;"|עוד כפלנו א' על ה' השני התחתון עלו ה' כתבנוהו ברביעית‫<ref group=I>כפלנו: Mo30; P1029 כפול; P1050; III נכפול<br> |
| + | א‫': Mo30; P1029 א' העליון<br> | ||
| + | ה‫': P1029 הה‫'<br> | ||
| + | השני: Lo27153 השנית; Mo30; P1029 om.<br> | ||
| + | התחתון: Mo30; P1029 מהטור התחתון; P1050 om.<br> | ||
| + | עלו: P1029 יעלו<br> | ||
| + | ה‫': P1050 ג"כ ה‫'<br> | ||
| + | כתבנוהו: Mo30; P1050 כתבנו; P1029 כתבהו<br> | ||
| + | ברביעית: Mo30; P1029 ברביעית תחת הב‫'</ref> | ||
|- | |- | ||
| | | | ||
:*We also multiply the upper 1 by the bottom 3; the result is 3. We write it in the fifth [rank] after 2. | :*We also multiply the upper 1 by the bottom 3; the result is 3. We write it in the fifth [rank] after 2. | ||
| − | |style="text-align:right;"|עוד כפלנו<ref group=I>כפלנו: Mo30; P1029 כפול; P1050; III נכפול< | + | |style="text-align:right;"|עוד כפלנו א' העליון על ג' התחתון היו ג' כתבנוהו בחמישית אחר ב'‫<ref group=I>כפלנו: Mo30; P1029 כפול; P1050; III נכפול<br> |
| + | א‫': Mo30 הא‫'<br> | ||
| + | התחתון: Mo30 מהטור העליון; P1029 מהטור התחתון; P1050 om.<br> | ||
| + | היו: Mo30; P1050; III עלו; P1029 יעלו<br> | ||
| + | כתבנוהו: Mo30; P1029 כתבהו; P1050 כתבנו<br> | ||
| + | בחמישית: P1029 בה‫'<br> | ||
| + | ב‫': Mo30; P1029 הב‫'<br> | ||
| + | אחר ב‫': P1050; III om.</ref> | ||
|- | |- | ||
| | | | ||
| Line 886: | Line 1,831: | ||
| | | | ||
:We add up all these numbers: all that is of the same rank together and what exceeds over ten or equal to ten, write it after that rank. | :We add up all these numbers: all that is of the same rank together and what exceeds over ten or equal to ten, write it after that rank. | ||
| − | |style="text-align:right;"|חברנו<ref group=I>חברנו: Mo30; P1029 חבר< | + | |style="text-align:right;"|חברנו כל אלו המספרים כל מה שהוא ממעלה אחת יחד הכל ומה שיעלה יותר מעשרה או עשרה כתבהו אחר המעלה ההיא‫<ref group=I>חברנו: Mo30; P1029 חבר<br> |
| + | אלו: Lo27153 <s>אלה</s> אילו; P1029; W152 אלה<br> | ||
| + | כל: Mo30 וכל; P1029 לכל<br> | ||
| + | ממעלה: Mo30; P1029 במעלה<br> | ||
| + | יחד: Mo30; P1029 תחבר; P1050 <sup>תחבר</sup> יחד<br> | ||
| + | או עשרה: P1029 om.<br> | ||
| + | כתבהו: Lo27153 כתבנוהו</ref> | ||
|- | |- | ||
| | | | ||
:The total product is 45 thousand and 85. | :The total product is 45 thousand and 85. | ||
| − | |style="text-align:right;"|ויעלה<ref group=I>ויעלה: Mo30; P1029 ותמצא שיעלה; III ועלה< | + | |style="text-align:right;"|ויעלה המחובר מ"ה אלפים ופ"ה‫<ref group=I>ויעלה: Mo30; P1029 ותמצא שיעלה; III ועלה<br> |
| + | ופ"ה: Mo30 ופ"ה למאזנים עשה כמו שידעת. נשלם השער הראשון; P1029 ופ"ה כזו הצורה וכלל זו הצורה כלומר המבוקש ממנה ה'ח'0'ה'ד' והמאזנים עשה כמו שידעת</ref> | ||
|- | |- | ||
|P1050 marg. another method | |P1050 marg. another method | ||
| Line 903: | Line 1,855: | ||
|style="text-align:right;"|עו' תשוב תכפול ב' עם ה' עלו י' והיה ראוי לכותבו תחת העשרות בשורה אחרת תחת הראשונה ולפי שהו' ר"ל י' עשרות שהם מאה כתו' עגול במדרגת העשרו' ושמור אחד שהוא מאה<br> | |style="text-align:right;"|עו' תשוב תכפול ב' עם ה' עלו י' והיה ראוי לכותבו תחת העשרות בשורה אחרת תחת הראשונה ולפי שהו' ר"ל י' עשרות שהם מאה כתו' עגול במדרגת העשרו' ושמור אחד שהוא מאה<br> | ||
וכפול ב' על ה' השני עלו י' וא' שהיה שמור הנה י"א כתו' א' במדרגת המאות ובידך שמור א' שהוא אלף<br> | וכפול ב' על ה' השני עלו י' וא' שהיה שמור הנה י"א כתו' א' במדרגת המאות ובידך שמור א' שהוא אלף<br> | ||
| − | עו' כפול ב' על ג' הנה הם ו' וא' הנה ז' כתו' ו' במדרגת האלפי' | + | עו' כפול ב' על ג' הנה הם ו' וא' הנה ז' כתו' ו' במדרגת האלפי‫' |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|עוד כפול א' על <s>ע</s> ה' וכתו' במדרגת המאות ה'<br> | + | |style="text-align:right;"|עוד כפול א' על <s>ע</s> ה' וכתו' במדרגת המאות ה‫'<br> |
| − | וא' על ה' השני וכתו' במדרגת האלפי' ה'<br> | + | וא' על ה' השני וכתו' במדרגת האלפי' ה‫'<br> |
| − | וכפול א' על ג' וכתו' במדרגת העשרת אלפי' ג'<br> | + | וכפול א' על ג' וכתו' במדרגת העשרת אלפי' ג‫'<br> |
וראה ועשה | וראה ועשה | ||
|- | |- | ||
| Line 926: | Line 1,878: | ||
|- | |- | ||
|<span style=color:green>Definition of a number:</span> {{#annot:definition|35|E7cZ}}Know that that every number is a sum of units. | |<span style=color:green>Definition of a number:</span> {{#annot:definition|35|E7cZ}}Know that that every number is a sum of units. | ||
| − | |style="text-align:right;"|דע כי<ref group=II>כי: V397 om.</ref> | + | |style="text-align:right;"|דע כי כל חשבון הוא חברת האחדים{{#annotend:E7cZ}}‫<ref group=II>כי: V397 om.</ref> |
|- | |- | ||
!<span style=color:green>One</span> | !<span style=color:green>One</span> | ||
| Line 932: | Line 1,884: | ||
|- | |- | ||
|One alone does not assume any change, no increase and no division. | |One alone does not assume any change, no increase and no division. | ||
| − | |style="text-align:right;"|והאחד<ref group= | + | |style="text-align:right;"|והאחד לבדו <div id="one_does_not_assume_change">לא יקבל שנוי</div>‫<ref group=note>[[Excerpts_Attributed_to_Immanuel_Bonfils#Does_not_assume_any_change|<span style=color:blue>commentary: does not assume any change</span>]]</ref> <div id="one_does_not_assume_increase">ולא רבוי</div>‫<ref group=note>[[Excerpts_Attributed_to_Immanuel_Bonfils#Does_not_assume_any_increase|<span style=color:blue>commentary: does not assume any increase</span>]]</ref> <div id="one_does_not_assume_division">ולא חלוק</div>‫<ref group=note>[[Excerpts_Attributed_to_Immanuel_Bonfils#Does_not_assume_any_division|<span style=color:blue>commentary: does not assume any division</span>]]</ref> |
| + | <ref group=II>והאחד: Mo30; P1051 רק האחד<br> | ||
| + | שנוי: Mo30 לא שנוי<br> | ||
| + | ולא: O187 לא</ref> | ||
|- | |- | ||
|It is the cause of every increase, change and division. | |It is the cause of every increase, change and division. | ||
| − | |style="text-align:right;"|והוא סבת כל<ref group= | + | |style="text-align:right;"|<div id="one_is_the_cause_of_increase_and_change">והוא סבת כל רבוי ושנוי וחלוק</div>‫<ref group=note>[[Excerpts_Attributed_to_Immanuel_Bonfils#It_is the_cause_of_any_change|<span style=color:blue>commentary: it is the cause of any change</span>]]</ref>‫<ref group=II>כל: V398 om.<br> |
| + | ושנוי: P1051 om.</ref> | ||
|- | |- | ||
| − | |One is eternal | + | |One is eternal. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<div id="one_is_eternal">והאחד קדמון לבדו</div>‫<ref group=note>[[פירוש_אנונימי_לספר_המספר#One_is_eternal|<span style=color:blue>commentary: one is eternal</span>]]</ref> |
| + | |- | ||
| + | |Every number created through it. | ||
| + | |style="text-align:right;"|וכל חשבון מתחדש בעבורו | ||
|- | |- | ||
| | | | ||
| Line 946: | Line 1,905: | ||
| | | | ||
:It does with one side what every [other] number does with its two sides. | :It does with one side what every [other] number does with its two sides. | ||
| − | |style="text-align:right;"|והוא יעשה<ref group=II>יעשה: V398 שיעשה< | + | |style="text-align:right;"|והוא יעשה בפאה אחת מה שיעשה כל חשבון בשתי פאותיו‫<ref group=II>יעשה: V398 שיעשה<br> |
| + | אחת: P1051; V398 האחת</ref> | ||
|- | |- | ||
| | | | ||
:For two is the preceding side for the number three and four is the other following side, and the sum of the two sides is 6, which is double the number three, and so for every number. | :For two is the preceding side for the number three and four is the other following side, and the sum of the two sides is 6, which is double the number three, and so for every number. | ||
| − | |style="text-align:right;"|כי שנים | + | |style="text-align:right;"|כי שנים לחשבון שלשה ה{{#annot:term|327|gUdN}}פאה{{#annotend:gUdN}} האחת שהיא לפניו וארבעה הפאה האחרת שהיא אחריו ושתי הפאות המחוברות ששה שהם כפל שלשה וככה כל מספר‫<ref group=II>שנים: Mo30 om.; O187 שתים<br> |
| + | שלשה: P1051 השלשה<br> | ||
| + | הפאה: O187; V397 הוא הפאה<br> | ||
| + | וארבעה הפאה: Mo30 ארבעה והפאה; P1051 שהיא הפאה<br> | ||
| + | ושתי: Mo30 שתים וב‫'<br> | ||
| + | המחוברות: Mo30 מחוברות<br> | ||
| + | שהם: Mo30 והוא; P1051</ref> | ||
|- | |- | ||
| | | | ||
:But the one has no side that precedes it and following it is one side, which is two, and it is double the one. | :But the one has no side that precedes it and following it is one side, which is two, and it is double the one. | ||
| − | |style="text-align:right;"|והנה האחד<ref group=II>האחד: O187; V397 אחד< | + | |style="text-align:right;"|והנה האחד אין לפניו פאה ואחריו פאה אחת שהיא שנים והם כפל האחד‫<ref group=II>האחד: O187; V397 אחד<br> |
| + | לפניו: P1051 לו marg. לפניו<br> | ||
| + | לפניו פאה ואחריו: O187 לפניו פאה ולאחריו; V397 פאה לפניו ולאחריו<br> | ||
| + | שנים: Mo30 שתים<br> | ||
| + | האחד: Mo30 אחד</ref> | ||
|- | |- | ||
|Now I shall talk about every number that has whole integers without fractions. | |Now I shall talk about every number that has whole integers without fractions. | ||
| − | |style="text-align:right;"|ועתה אדבר על כל חשבון שיש לו אחדים שלמים בלי<ref group=II>בלי: Mo30 ולא</ref> | + | |style="text-align:right;"|ועתה אדבר על כל חשבון שיש לו אחדים שלמים בלי שבר‫<ref group=II>בלי: Mo30 ולא</ref> |
|- | |- | ||
!<span style=color:green>Sexagesimal Fractions</span> | !<span style=color:green>Sexagesimal Fractions</span> | ||
| Line 963: | Line 1,933: | ||
|- | |- | ||
|<span style=color:green>12 zodiac signs:</span> Know that the astrologers have divided the celestial sphere into twelve parts. | |<span style=color:green>12 zodiac signs:</span> Know that the astrologers have divided the celestial sphere into twelve parts. | ||
| − | |style="text-align:right;"|ודע כי חכמי המזלות חלקו את<ref group=II>את: Mo30; P1051; V398 om.< | + | |style="text-align:right;"|ודע כי חכמי המזלות חלקו את הגלגל על שנים עשר חלקים‫<ref group=II>את: Mo30; P1051; V398 om.<br> |
| + | על שנים עשר: O187; P1051; V397 לי"ב</ref> | ||
|- | |- | ||
| | | | ||
::They did this because the solar year has 12 lunar months and because there is no number, smaller than 12, that has as many parts as it, for it has whole integers in its half, its third, its quarter, its sixth and its half of one-sixth. | ::They did this because the solar year has 12 lunar months and because there is no number, smaller than 12, that has as many parts as it, for it has whole integers in its half, its third, its quarter, its sixth and its half of one-sixth. | ||
| − | |style="text-align:right;"|ועשו זה בעבור ששנת השמש י"ב<ref group=II>י"ב: O187 היא שנים עשר; V397 היא י"ב< | + | |style="text-align:right;"|<div id="they_did_this"></div>ועשו זה בעבור ששנת השמש י"ב חדשי הלבנה ואין חשבון קטן מי"ב שיש לו חלקים רבים כמוהו כי יש לו אחדים שלמים בחציו ושלישיתו ורביעיתו וששיתו וחצי ששיתו‫<ref group=note>P1051 marg. אינו מן הספר; V398 marg. ו' ד' ג' ב‫'</ref>‫<ref group=II>י"ב: O187 היא שנים עשר; V397 היא י"ב<br> |
| + | חדשי: V397 חדש<br> | ||
| + | הלבנה: P1051 לבנה<br> | ||
| + | חשבון: O187; V397 בחשבון<br> | ||
| + | רבים: V397 om.<br> | ||
| + | אחדים: P1051 <s>חלקים רבים אחדי</s><br> | ||
| + | שלמים: Mo30; P1051 שלמים בלי שבר<br> | ||
| + | ושלישיתו: Mo30p; V398 ובשלישיתו<br> | ||
| + | ורביעיתו: Mo30 וברביעיתו<br> | ||
| + | וחצי ששיתו: Mo30; V398 om.</ref>‫<ref group=note>[[פירוש_אנונימי_לספר_המספר#They_did_this|<span style=color:blue>commentary: they did this</span>]]</ref> | ||
|- | |- | ||
|<span style=color:green>degrees:</span> They divided each sign to thirty degrees. | |<span style=color:green>degrees:</span> They divided each sign to thirty degrees. | ||
| − | |style="text-align:right;"|וחלקו המזל לשלשים<ref group=II>לשלשים: O187 ל'</ref> | + | |style="text-align:right;"|<div id="they_divided_each_sign_to_thirty"></div>וחלקו המזל לשלשים מעלות‫<ref group=II>לשלשים: O187 ל‫'</ref> |
|- | |- | ||
| | | | ||
::Because this number has more whole units than 12; for it has one-half, one-third, one-fifth, one-sixth and one-tenth. | ::Because this number has more whole units than 12; for it has one-half, one-third, one-fifth, one-sixth and one-tenth. | ||
| − | |style="text-align:right;"|כי זה המספר יש לו אחדים שלמים יותר מי"ב כי יש לו חצי ושלישית וחמישית וששית<ref group=II>וששית: Mo30 om.<br>וחמישית וששית: P1051 וששית וחמישית</ref> | + | |style="text-align:right;"|כי זה המספר יש לו אחדים שלמים יותר מי"ב כי יש לו חצי ושלישית וחמישית וששית ועשירית‫<ref group=note>V398 marg. ט"ו י' ו' ה' ג‫'</ref>‫<ref group=II>וששית: Mo30 om.<br> |
| + | וחמישית וששית: P1051 וששית וחמישית</ref>‫<ref group=note>[[פירוש_אנונימי_לספר_המספר#They_divided_each_sign_to_thirty_degrees.2C_because_this_number_has_more_whole_units_than_12.3B_for_it_has_one-half.2C_one-third_etc.|<span style=color:blue>commentary: they divided each sign to thirty</span>]]</ref> | ||
|- | |- | ||
| | | | ||
::So, the number of degrees of the celestial sphere is 360. | ::So, the number of degrees of the celestial sphere is 360. | ||
| − | |style="text-align:right;"|והנה עלה מספר<ref group=II>מספר: V398 המספר< | + | |style="text-align:right;"|והנה עלה מספר מעלות הגלגל ש"ס‫<ref group=II>מספר: V398 המספר<br> |
| + | מעלות: V397 om.<br> | ||
| + | הגלגל: V398 om.<br> | ||
| + | ש"ס: O187 לש"ס</ref> | ||
|- | |- | ||
| | | | ||
::This number has one-half, one-third, a quarter, one-fifth, one-sixth, one-eighth, one-ninth and one-tenth only the seventh is missing. | ::This number has one-half, one-third, a quarter, one-fifth, one-sixth, one-eighth, one-ninth and one-tenth only the seventh is missing. | ||
| − | |style="text-align:right;"|וזה המספר<ref group=II>וזה המספר: O187 וזה המספר קרוב לימות שנת החמה וזה המספר; P1051 וזה המספר יש לו קרוב לימות החמה; V397 וזה המספר לימי שנות החמה וזה המספר< | + | |style="text-align:right;"|וזה המספר יש לו חצי ושלישית ורביעית וחמישית וששית ושמינית ותשיעית ועשירית והנה לא יחסר לו רק השביעית‫<ref group=note>V398 marg. ק"פ ק"כ צ' ע"ב ס' מ"ה מ' ל"ו</ref>‫<ref group=II>וזה המספר: O187 וזה המספר קרוב לימות שנת החמה וזה המספר; P1051 וזה המספר יש לו קרוב לימות החמה; V397 וזה המספר לימי שנות החמה וזה המספר<br> |
| + | יש: P1051 ויש<br> | ||
| + | ושלישית: Mo30 ושליש<br> | ||
| + | ורביעית: Mo30 ורביע<br> | ||
| + | וחמישית: Mo30 וחומש<br> | ||
| + | ועשירית: Mo30 ועשור<br> | ||
| + | והנה לא: Mo30 ולא<br> | ||
| + | רק: P1051 top</ref> | ||
|- | |- | ||
| | | | ||
::When you multiply this number by 7, the result is two thousand and 520, and this number includes all fractions up to tenths. | ::When you multiply this number by 7, the result is two thousand and 520, and this number includes all fractions up to tenths. | ||
| − | |style="text-align:right;"|וכאשר תכפול זה המספר | + | |style="text-align:right;"|וכאשר תכפול זה המספר על ז' יהיה העולה אלפים ותק"כ וזה החשבון כולל כל החלקים עד עשרה‫<ref group=II>המספר: V397 om.<br> |
| + | עשרה: O187 העשרה</ref> | ||
|- | |- | ||
| | | | ||
*When the astrologers multiply degrees by degrees, the product is degrees, which are whole units. | *When the astrologers multiply degrees by degrees, the product is degrees, which are whole units. | ||
| − | |style="text-align:right;"|והנה חכמי המזלות כאשר יכפלו מעלות<ref group=II>מעלות: V397 המעלות< | + | |style="text-align:right;"|והנה חכמי המזלות כאשר יכפלו מעלות על מעלות יהיה המחובר מעלות שהם אחדים שלמים‫<ref group=II>מעלות: V397 המעלות<br> |
| + | שהם: P1051; V397 שהן<br> | ||
| + | אחדים: O187; V397 כאחדים</ref> | ||
|- | |- | ||
| | | | ||
*Similarly, when they divide degrees by degrees, the quotient is degrees, which are whole units. | *Similarly, when they divide degrees by degrees, the quotient is degrees, which are whole units. | ||
| − | |style="text-align:right;"|וככה כאשר יחלקו<ref group=II>יחלקו: Mo30; P1051; V397 יתחלקו< | + | |style="text-align:right;"|וככה כאשר יחלקו מעלות על מעלות יהיה העולה בחלוק מעלות שהם אחדים שלמים‫<ref group=II>יחלקו: Mo30; P1051; V397 יתחלקו<br> |
| + | העולה בחלוק: Mo30; P1051 המחובר<br> | ||
| + | וככה ... שלמים: Mo30 marg.; V398 om.</ref> | ||
|- | |- | ||
| | | | ||
| Line 1,003: | Line 1,999: | ||
|- | |- | ||
|Now I shall give you a rule how to divide every number, whether it consists of one, two or many digits: | |Now I shall give you a rule how to divide every number, whether it consists of one, two or many digits: | ||
| − | |style="text-align:right;"|ועתה אתן לך כלל<ref group=II>כלל: P1051 <s>משל</s> כלל</ref> | + | |style="text-align:right;"|ועתה אתן לך כלל איך תחלק כל חשבון בין שיהיה אחד או שנים או מספרים רבים‫<ref group=II>כלל: P1051 <s>משל</s> כלל<br> |
| + | תחלק: O187 תסלק; V398 יתחלק</ref> | ||
| + | |- | ||
| + | |Write them in one row, each according to its rank. | ||
| + | |style="text-align:right;"|כתוב אותם בטור אחד <div id="each_according_to_its_rank">כל אחד כפי מעלתו</div>‫<ref group=note>[[פירוש_אנונימי_לספר_המספר#Each_according_to_its_rank|<span style=color:blue>anonymous commentary: each according to its rank</span>]]; [[Excerpts_Attributed_to_Immanuel_Bonfils#According_to_its_rank|<span style=color:blue>Bonfils commentary: each according to its rank</span>]]</ref>‫<ref group=II>אותם: V397 אותן<br> | ||
| + | כפי: O187; V397 לפי</ref> | ||
|- | |- | ||
| − | | | + | |Then, write the number by which you divide in another row, whether it consists of one or many digits, each according to its rank corresponding to each digit according to its rank in the top row, and leave a space between the upper row and the bottom row so that you can write a middle row in between them; whether the quotient consists of one or many digits, you place each one according to its rank. |
| − | |style="text-align:right;"|כתוב | + | |style="text-align:right;"|ואחר כך כתוב החשבון שתחלק עליו בטור אחר בין שיהיה מספר אחד או רבים כל אחד כפי מעלתו ויהיה כל חשבון לפי מעלתו כנגד כל חשבון כפי מעלתו בטור העליון וריוח תשים בין הטור העליון והטור השפל כדי שתוכל לכתוב טור אמצעי ביניהם בין שיהיה העולה מספר אחד או מספרים רבים כל אחד תשים כפי מעלתו‫<ref group=II>ואחר כך: Mo30 אח"כ<br> |
| + | אותם ... כתוב: V397 marg.<br> | ||
| + | בטור: O187 הטור<br> | ||
| + | אחר: O187; V397 האחר<br> | ||
| + | בטור אחר: P1051 om.<br> | ||
| + | שיהיה: Mo30 שהיה<br> | ||
| + | ואחר כך ... מעלתו: V398 om.<br> | ||
| + | חשבון: Mo30 החשבון<br> | ||
| + | ויהיה כל חשבון לפי מעלתו: P1051 om.; V398 marg. ויהיה כל חשבון לפי מעלתו בטור השפל<br> | ||
| + | כל: O187 top<br> | ||
| + | לפי מעלתו כנגד כל חשבון: Mo30 om.<br> | ||
| + | הטור: O187; V397 טור<br> | ||
| + | והטור: O187; V397 לטור<br> | ||
| + | אמצעי: Mo30 האמצעי; O187; V397 אחד<br> | ||
| + | ביניהם: O187; V397 בין שניהם<br> | ||
| + | תשים כפי מעלתו: V397 כפי מעלתו תשים</ref> | ||
|- | |- | ||
|The number by which you divide should be less than the number that you divide by it. | |The number by which you divide should be less than the number that you divide by it. | ||
| − | |style="text-align:right;"|וראוי להיות המספר שתחלק עליו פחות מהמספר המחולק ממנו | + | |style="text-align:right;"|<div id="the_divisor_should_be_less_than_the_dividend">וראוי להיות המספר שתחלק עליו פחות מהמספר המחולק ממנו</div> וזה הדבר הוא בחלוק השלמים‫<ref group=note>[[פירוש_אנונימי_לספר_המספר#The_number_by_which_you_divide_should_be_less_than_the_dividend|<span style=color:blue>anonymous commentary: the number by which you divide should be less than the dividend</span>]]; [[Excerpts_Attributed_to_Immanuel_Bonfils#Less_than_the_number|<span style=color:blue>Bonfils commentary: the divisor should be less than the dividend</span>]]</ref><ref group=II>ממנו: Mo30; P1051; V397 om.<br> |
| + | השלמים: V397 בשלמים</ref> | ||
|- | |- | ||
|But this is only for the division of integers, not for fractions, as I will explain with the help of God. | |But this is only for the division of integers, not for fractions, as I will explain with the help of God. | ||
| − | |style="text-align:right;"|ולא<ref group=II>ולא: Mo30 לא</ref> | + | |style="text-align:right;"|ולא כן בשברים כאשר אפרש בעזרת האל‫<ref group=II>ולא: Mo30 לא</ref> |
|- | |- | ||
|When you set up the rows, as I said, you start dividing by the last digit in the top row. | |When you set up the rows, as I said, you start dividing by the last digit in the top row. | ||
| − | |style="text-align:right;"|ובתקנך הטורים כאשר אמרתי תחל<ref group=II>תחל: V397 תחל<s>ק</s>< | + | |style="text-align:right;"|ובתקנך הטורים כאשר אמרתי תחל לחלק מהמספר האחרון שהוא בטור העליון‫<ref group=II>תחל: V397 תחל<s>ק</s><br> |
| + | בטור: V398 om.</ref> | ||
|- | |- | ||
|Divide it by the last number in the bottom row. | |Divide it by the last number in the bottom row. | ||
| − | |style="text-align:right;"|ותחלק אותו על המספר האחרון שהוא בטור<ref group=II>שהוא בטור: Mo30 שבטור< | + | |style="text-align:right;"|ותחלק אותו על המספר האחרון שהוא בטור השפל‫<ref group=II>שהוא בטור: Mo30 שבטור<br> |
| + | השפל: P1051 <s>העליון</s> השפל</ref> | ||
|- | |- | ||
|Consider the two numbers, even though they are decades, consider them as units. | |Consider the two numbers, even though they are decades, consider them as units. | ||
| − | |style="text-align:right;"|וחשוב שנים<ref group= | + | |style="text-align:right;"|וחשוב שנים המספרים אע"פ שהם כללים חשוב אותם כמו אחדים‫<ref group=note>P1051 marg. פי' ק' ואחר כך תמצא אמתתם במעלתם</ref>‫<ref group=II>שנים: V397 שני<br> |
| + | המספרים: Mo30 מס‫'; P1051 <sup>ה</sup>מספרים<br> | ||
| + | חשוב: P1051 חשב<br> | ||
| + | אותם: V398 אותו</ref> | ||
| + | |- | ||
| + | |The quotient: see how far the last digit of the bottom row is from the first digit, whether it is units or a zero, and as the number of the distance, return back. | ||
| + | |style="text-align:right;"|והעולה בחלוק ראה כמה מרחק המספר האחרון מהטור השפל מהמספר הראשון בין שיהיו בו אחדים או גלגל <div id="return_back_as_the_number_of_the_distance">וכפי מספר המרחק תשיב אחורנית</div>‫<ref group=note>P1051 marg. פי' ק' מהמספר האחרון שבטור העליון</ref><ref group=note>[[פירוש_אנונימי_לספר_המספר#Return_back_as_the_number_of_the_distance|<span style=color:blue>commentary: return back as the number of the distance</span>]]</ref>‫<ref group=II>המספר: V397 מספר<br> | ||
| + | העליון ותחלק ... מהטור השפל: Mo30 marg.<br> | ||
| + | שיהיו: V397 שיהיה<br> | ||
| + | תשיב: Mo30; P1051; V398 תשוב</ref> | ||
| + | |- | ||
| + | |Write the quotient there above the bottom row, which is beneath the top row. | ||
| + | |style="text-align:right;"|ושם תכתוב העולה בחלוק למעלה מהטור השפל שהוא למטה מהטור העליון | ||
|- | |- | ||
| − | | | + | |If a number that cannot be divided remains from the last digit, and [the division] has not yet reached the rank of the units, return the remaining number back to the preceding rank, which is lower than it. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<div id="if_a_number_that_cannot_be_divided_remains_from_the_last_digit">ואם ישאר במספר האחרון חשבון שלא נתחלק</div>‫<ref group=note>[[פירוש_אנונימי_לספר_המספר#If_a_number_that_cannot_be_divided_remains_from_the_last_digit|<span style=color:blue>commentary: if a number that cannot be divided remains from the last digit</span>]]</ref> <div id="not_reached_the_units">ולא הגיע למעלת האחדים</div>‫<ref group=note> [[פירוש_אנונימי_לספר_המספר#Has_not_yet_reached_the_rank_of_the_units|<span style=color:blue>anonymous commentary: has not yet reached the rank of the units</span>]]; [[Excerpts_Attributed_to_Immanuel_Bonfils#Did_not_reach_the_rank_of_the_units|<span style=color:blue>Bonfils commentary: has not yet reached the rank of the units</span>]]</ref> <div id="return_the_remaining_number_back_to_the_preceding_rank">השב אחורנית המספר הנשאר למעלה הראשונה שהיא פחותה ממנו</div>‫<ref group=note>[[פירוש_אנונימי_לספר_המספר#Return_the_remaining_number_back_to_the_preceding_rank.2C_which_is_lower_than_it|<span style=color:blue>commentary: return the remaining number back to the preceding rank which is lower than it</span>]]</ref>‫<ref group=II>האחרון: V397 אחרון<br> |
| + | האחדים: P1051; V398 אחדים<br> | ||
| + | למעלה: Mo30 ולמעלה</ref> | ||
|- | |- | ||
| − | | | + | |Consider each unit as ten. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וחשוב כל אחד עשרה‫<ref group=II>כל: Mo30 ככל</ref> |
|- | |- | ||
|Then, divide by the number by which you divide, and write the quotient back from the first rank you wrote, before the first quotient. | |Then, divide by the number by which you divide, and write the quotient back from the first rank you wrote, before the first quotient. | ||
| − | |style="text-align:right;"|ואחר כך חלק על המספר<ref group=II>המספר: V398 מספר</ref> | + | |style="text-align:right;"|ואחר כך חלק על המספר שחלקת עליו והעולה בחלוק תכתוב אותו אחורנית מהמעלה הראשונה שכתבת לפני מה שעלה בחלוק בראשונה‫<ref group=II>המספר: V398 מספר<br> |
| + | תכתוב אותו: Mo30 תכתבהו<br> | ||
| + | לפני: Mo30; V397 om.</ref> | ||
| + | |- | ||
| + | |Proceed always like this until you reach a number that is smaller than the divisor. | ||
| + | |style="text-align:right;"|ככה תעשה תמיד עד שתגיע אל המספר שהוא פחות מהמחולק עליו‫<ref group=II>שתגיע: V397 שישיג; V398 שיגיע<br> | ||
| + | המספר: Mo30; P1051; V398 מספר<br> | ||
| + | מהמחולק עליו: Mo30 מהמחלק</ref> | ||
| + | |- | ||
| + | |Write the remainder above the top row according to its rank. | ||
| + | |style="text-align:right;"|<div id="write_the_remainder_above_the_top_row_according_to_its_rank">ואותו הנשאר תכתבנו למעלה מהטור העליון כפי מעלתו</div>‫<ref group=note>[[פירוש_אנונימי_לספר_המספר#Write_the_remainder_above_the_top_row_according_to_its_rank|<span style=color:blue>commentary: write the remainder above the top row according to its rank</span>]]</ref>‫<ref group=II>תכתבנו: Mo30 תכתבהו<br> | ||
| + | מהטור: Mo30; P1051 מן הטור</ref> | ||
|- | |- | ||
| − | | | + | |In the fifth chapter I will explain what you should do with it. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<div id="in_the_fifth_chapter"></div>ובשער החמישי אפרש לך מה שתעשה ממנו‫<ref group=note>[[פירוש_אנונימי_לספר_המספר#In_the_fifth_chapter|<span style=color:blue>commentary: in the fifth chapter</span>]]</ref>‫<ref group=II>החמישי: P1051 השלישי<br> |
| + | שתעשה: Mo30; P1051 תעשה</ref> | ||
|- | |- | ||
| | | | ||
*{{#annot:9000÷70|157|ZS2i}}Example: we wish to divide 9 thousands by 70. | *{{#annot:9000÷70|157|ZS2i}}Example: we wish to divide 9 thousands by 70. | ||
:<math>\scriptstyle9000\div70</math> | :<math>\scriptstyle9000\div70</math> | ||
| − | |style="text-align:right;"|<big>דמיון</big | + | |style="text-align:right;"|<big>דמיון</big> בקשנו לחלק ט' אלפים על ע‫'{{#annotend:ZS2i}}‫<ref group=II>דמיון: Mo30 דמיון זה</ref> |
|- | |- | ||
| | | | ||
| Line 1,098: | Line 2,143: | ||
|- | |- | ||
| | | | ||
| − | :*We put 70 in the bottom row according to its ranks and consider all as units. We give it 1 | + | :*We put 70 in the bottom row according to its ranks and consider all as units. We give it 1. |
| − | |style="text-align:right;"|הנה נשים ע'<ref group=II>ע': Mo30 ז'; P1051 ט'< | + | |style="text-align:right;"|הנה נשים ע' בטור השפל כפי מעלתו ונחשוב כי הכל אחדים <div id="we_give_it_1">והנה נתן לו א‫'</div>‫<ref group=note>[[פירוש_אנונימי_לספר_המספר#We_give_it_1|<span style=color:blue>commentary: we give it 1</span>]]</ref>‫<ref group=II>ע‫': Mo30 ז‫'; P1051 ט‫'<br> |
| + | השפל: Mo30 שפל כפי מעלתו וט' בטור עליון; P1051 העליון; V398 שפל<br> | ||
| + | והנה: P1051 הנה<br> | ||
| + | נתן לו: P1051 נתתי לך</ref> | ||
| + | |- | ||
| + | | | ||
| + | ::We write it in the second rank back from the last digit of the first row, because the 70 is in the second rank in the lower row and we write it in the middle. We are left with two. | ||
| + | |style="text-align:right;"|ונכתבנו במעלה השנית אחורנית מהמספר האחרון שהוא בטור הראשון כי ע' הוא שני לטור השפל‫<ref group=note>Mo30 marg. ר"ל כשנתחיל מהאחדים</ref> ונכתבנו באמצע ונשארו לנו שנים‫<ref group=II>ונכתבנו: Mo30 ונכתבהו; V397 וכתבנו ונכתבנו<br> | ||
| + | השנית: V397 שנית<br> | ||
| + | מהמספר: Mo30 ממספר<br> | ||
| + | הראשון: Mo30; P1051; V398 העליון<br> | ||
| + | ונכתבנו: Mo30 ונכתבהו<br> | ||
| + | לנו: P1051 om.</ref> | ||
|- | |- | ||
| | | | ||
:*We return it back, so it is twenty. We divide [them] by 7, we give it 2 and write it back before what was written first; we are left with 6. | :*We return it back, so it is twenty. We divide [them] by 7, we give it 2 and write it back before what was written first; we are left with 6. | ||
| − | |style="text-align:right;"|נשיבם אחורנית והם עשרים נחלק | + | |style="text-align:right;"|נשיבם אחורנית והם עשרים נחלק על ז' והנה נתן לו ב' ונכתוב אותו אחורנית לפני הנכתב בראשונה‫<ref group=note>P1051 marg. פי' ק' פי' שהוא במעלה שנית אחורנית מהמספר המחלק</ref> ונשארו לנו ו‫'‫<ref group=II>נחלק: Mo30 נחלקם<br> |
| + | והנה נתן: Mo30 ונתן<br> | ||
| + | לו: P1051 לנו</ref> | ||
|- | |- | ||
| | | | ||
:*We return it back, so it is sixty. We divide them by 7, we give it 8. Write it back. | :*We return it back, so it is sixty. We divide them by 7, we give it 8. Write it back. | ||
| − | |style="text-align:right;"|נשיבהו<ref group= | + | |style="text-align:right;"|נשיבהו אחורנית יהיו ששים נחלקנו על ז' נתן לו ח' תכתבהו אחורנית‫<ref group=note>P1051 marg. פי' לפני הנכתב שנית שהוא במעלה שנית אחורנית מהמספר המחלק</ref>‫<ref group=II>נשיבהו: V397 נשיבם<br> |
| + | יהיו: Mo30 ויהיו<br> | ||
| + | נחלקנו: Mo30 נחלקהו; V398 נחלקם<br> | ||
| + | לו: P1051 לנו<br> | ||
| + | נתן לו: Mo30 ויהיו<br> | ||
| + | תכתבהו: Mo30 ונכתבהו; P1051; V398 ונכתבנו</ref> | ||
|- | |- | ||
| | | | ||
:We are left with 4, which is in the second rank, so it is 40 and the divisor is greater than it. | :We are left with 4, which is in the second rank, so it is 40 and the divisor is greater than it. | ||
| − | |style="text-align:right;"|ונשארו לנו ד'<ref group=II>ד': V397 ה'< | + | |style="text-align:right;"|ונשארו לנו ד' והם במעלה השנית והם מ' והמספר המחולק עליו גדול ממנו‫<ref group=II>ד‫': V397 ה‫'<br> |
| + | מ‫': V397 <s>מ‫'</s> <sup>נ‫'</sup><br> | ||
| + | עליו: V398 om.<br> | ||
| + | המחולק עליו: Mo30 החולק<br> | ||
| + | והמספר המחולק עליו גדול ממנו: P1051 om.</ref> | ||
| + | |- | ||
| + | |If the divisor as units is greater than the last digit in the top row, return it back and calculate from this position. | ||
| + | |style="text-align:right;"|ואם היה המספר באחדים המחולק עליו גדול מהמספר האחרון בטור העליון תשיבהו אחורנית <div id="calculate_from_this_position">ותחשוב מאותו מקום</div>‫<ref group=note>[[פירוש_אנונימי_לספר_המספר#Calculate_from_this_position|<span style=color:blue>commentary: calculate from this position</span>]]</ref>‫<ref group=II>באחדים: Mo30 מאחדים<br> | ||
| + | היה המספר באחדים: P1051 om.<br> | ||
| + | ותחשוב: V397 om.<br> | ||
| + | מאותו מקום: Mo30 אותו המקום</ref> | ||
| + | |- | ||
| + | |Return it back according to the distance of the divisor. | ||
| + | |style="text-align:right;"|<div id="according_to_the_distance_of_the_divisor">וכפי מרחק המספר המחולק עליו תשיב אחורנית</div>‫<ref group=note>[[פירוש_אנונימי_לספר_המספר#According_to_the_distance_of_the_divisor|<span style=color:blue>commentary: according to the distance of the divisor</span>]]</ref>‫<ref group=II>מרחק: P1051 המרחק<br> | ||
| + | תשיב: Mo30 תשוב<br> | ||
| + | אחורנית: P1051 אחורנית marg. וחשוב כל אחד עשרה</ref> | ||
|- | |- | ||
| − | | | + | |Proceed according to the rule. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ועשה כמשפט‫<ref group=II>ועשה: V397 ותעשה</ref> |
|- | |- | ||
| | | | ||
*{{#annot:20000÷90|157|dbse}}Example: we wish to divide 20 thousands by 90. | *{{#annot:20000÷90|157|dbse}}Example: we wish to divide 20 thousands by 90. | ||
:<math>\scriptstyle20000\div90</math> | :<math>\scriptstyle20000\div90</math> | ||
| − | |style="text-align:right;"|<big>דמיון</big> בקשנו | + | |style="text-align:right;"|<big>דמיון</big> בקשנו לחלק כ' אלף על צ‫'{{#annotend:dbse}}‫<ref group=II>בקשנו: V397 רצינו</ref> |
|- | |- | ||
| | | | ||
| Line 1,180: | Line 2,259: | ||
| | | | ||
:*Since the number 9 is greater than 2, we return it back, so it is 20. We divide it by 9; the result is 2. We write it back in the third rank, which is the second to the digit that we divided; 2 remain. | :*Since the number 9 is greater than 2, we return it back, so it is 20. We divide it by 9; the result is 2. We write it back in the third rank, which is the second to the digit that we divided; 2 remain. | ||
| − | |style="text-align:right;"|והנה<ref group=II>והנה: Mo30; V398 הנה< | + | |style="text-align:right;"|והנה בעבור כי מספר ט' גדול מב' נשיבהו אחורנית והם כ' נחלקם על ט' והנה ב' נכתבנו במעלה השלישית אחורנית שהיא שנית לחשבון שחלקנו ממנו ונשארו ב‫'‫<ref group=II>והנה: Mo30; V398 הנה<br> |
| + | מספר: V397 משפט<br> | ||
| + | נשיבהו: Mo30 נשיבם<br> | ||
| + | אחורנית: V397 אחורנית במעלה הרביעית<br> | ||
| + | והם: V397 והנה<br> | ||
| + | נחלקם: Mo30 ונחלקם; P1051; V397 נחלק<br> | ||
| + | והנה: Mo30 ונתן לו marg. והנה; P1051 והנם; V398 ויהיו<br> | ||
| + | נכתבנו: Mo30; V397 ונכתבנו<br> | ||
| + | ב' ... ממנו: Mo30 marg.<br> | ||
| + | ב‫': V397 <s>עשרים נחלק</s> <sup>ב‫'</sup></ref> | ||
|- | |- | ||
| | | | ||
:*We return them back to the third rank, so they are 20. We divide them by 9; the result is 2, and 2 remain. | :*We return them back to the third rank, so they are 20. We divide them by 9; the result is 2, and 2 remain. | ||
| − | |style="text-align:right;"|נשיבם<ref group=II>נשיבם: Mo30 עוד נשיבם; P1051 ונשיבם; V398 נשיב ב'< | + | |style="text-align:right;"|נשיבם אחורנית במעלה השלישית והם כ' נחלקם על ט' והנה ב' ונשארו ב‫'‫<ref group=II>נשיבם: Mo30 עוד נשיבם; P1051 ונשיבם; V398 נשיב ב‫'<br> |
| + | במעלה השלישית: Mo30 om.<br> | ||
| + | אחורנית שהיא שנית ... במעלה השלישית: P1051 marg.<br> | ||
| + | והם: P1051 הם<br> | ||
| + | נחלקם: P1051 נחלק<br> | ||
| + | נחלקם על ט‫': Mo30 om.<br> | ||
| + | והנה: Mo30 ונתן לו; P1051; V398 והנם<br> | ||
| + | ונשארו: Mo30 נשארו עוד; P1051 נשארו<br> | ||
| + | נשיבם ... ב‫': V397 om.</ref> | ||
|- | |- | ||
| | | | ||
:*We return them back to the second rank, they are twenty. We divide them by 9; the result is and 2 remain, which are twenty, because they are in the second rank in the top row. This number is smaller than our number, so we write back a zero, because no units resulted, and it cannot be taken out completely. | :*We return them back to the second rank, they are twenty. We divide them by 9; the result is and 2 remain, which are twenty, because they are in the second rank in the top row. This number is smaller than our number, so we write back a zero, because no units resulted, and it cannot be taken out completely. | ||
| − | |style="text-align:right;"|נשיבם אחורנית במעלה השנית<ref group=II>השנית: Mo30 השנית <sup>הג'</sup>< | + | |style="text-align:right;"|נשיבם אחורנית במעלה השנית והם עשרים נחלק על ט' והנם ב' ונשארו ב' שהם עשרים כי הם במעלה השנית בטור העליון וזה המספר פחות ממספרנו על כן נכתוב גלגל אחורנית כי לא עלו אחדים <div id="not_gone_completely">כי לא יצא לחוץ</div>‫<ref group=note>[[Excerpts_Attributed_to_Immanuel_Bonfils#Not_gone_completely|<span style=color:blue>commentary: not gone completely</span>]]</ref><ref group=II>השנית: Mo30 השנית <sup>הג‫'</sup><br> |
| + | נחלק על ט‫': Mo30 om.<br> | ||
| + | והנם: Mo30 ונתן לו<br> | ||
| + | והנם ב‫': V397 om.<br> | ||
| + | ונשארו: Mo30; P1051 נשארו<br> | ||
| + | כי הם: V397 שהם<br> | ||
| + | כי הם ... העליון: Mo30 om.<br> | ||
| + | וזה המספר: Mo30 והוא<br> | ||
| + | ממספרנו: Mo30 מהחולק; P1051 ממספר ט‫'<br> | ||
| + | על: Mo30 ועל<br> | ||
| + | עלו אחדים: V398 אחדים הם</ref> | ||
|- | |- | ||
|If there is a zero in one of the positions and you cannot divide by the divisor, return it back from the higher [rank]. | |If there is a zero in one of the positions and you cannot divide by the divisor, return it back from the higher [rank]. | ||
| − | |style="text-align:right;"|ואם היה גלגל<ref group= | + | |style="text-align:right;"|<div id="if_there_is_a_zero_in_one_of_the_positions"></div>ואם היה גלגל באחד המקומות ולא תוכל לחלק על המספר המחולק השב אחורנית מהגבוה ממנו‫<ref group=note>[[פירוש_אנונימי_לספר_המספר#If_there_is_a_zero_in_one_of_the_positions|<span style=color:blue>commentary: if there is a zero in one of the positions</span>]]</ref>‫<ref group=II>גלגל: Mo30 הגלגל<br> |
| + | המקומות: V397 <sup>מ</sup>המקומות<br> | ||
| + | ולא: Mo30 לא<br> | ||
| + | תוכל: P1051 נוכל<br> | ||
| + | המחולק: Mo30 om.<br> | ||
| + | מהגבוה: P1051 מגבוה</ref> | ||
|- | |- | ||
| | | | ||
*{{#annot:4032÷30|157|wcHu}}Example: we wish to divide 4 thousands and 32 by 30. | *{{#annot:4032÷30|157|wcHu}}Example: we wish to divide 4 thousands and 32 by 30. | ||
:<math>\scriptstyle4032\div30</math> | :<math>\scriptstyle4032\div30</math> | ||
| − | |style="text-align:right;"|<big>דמיון</big> בקשנו<ref group=II>בקשנו: Mo30 נרצה< | + | |style="text-align:right;"|<big>דמיון</big> בקשנו לחלק ד' אלפים ול"ב על שלשים{{#annotend:wcHu}}‫<ref group=II>בקשנו: Mo30 נרצה<br> |
| + | בקשנו לחלק: P1051 חלקנו; V398 om.<br> | ||
| + | בקשנו ... שלשים: V397 om.<br> | ||
| + | ד' אלפים ול"ב על שלשים: V398 marg.</ref> | ||
|- | |- | ||
| | | | ||
| Line 1,253: | Line 2,367: | ||
| | | | ||
:*We divide 4 by 3; we get 1. We write it back in the rank of the hundreds, because it is second to it; we are still left with 1. | :*We divide 4 by 3; we get 1. We write it back in the rank of the hundreds, because it is second to it; we are still left with 1. | ||
| − | |style="text-align:right;"|חלקנו ד' על ג'<ref group=II>חלקנו ד' על ג': P1051 om.< | + | |style="text-align:right;"|חלקנו ד' על ג' ועלה בידינו א' וכתבנוהו אחורנית במעלת המאות כי הוא שני לו נשאר לנו עוד א‫'‫<ref group=note>P1051 marg. ק' פי' על ד‫'</ref>‫<ref group=II>חלקנו ד' על ג‫': P1051 om.<br> |
| + | א‫': V398 אחד marg. ונשאר אחד<br> | ||
| + | במעלת: V397 במעלה<br> | ||
| + | וכתבנוהו ... שני לו: Mo30 om.<br> | ||
| + | נשאר: Mo30 ונשארו<br> | ||
| + | לנו: Mo30 om.<br> | ||
| + | עוד: Mo30; V398 om.</ref> | ||
|- | |- | ||
| | | | ||
:*We return it back to the rank of the hundreds; so, they are 10. We divide them by 3; the quotient is 3, 1 is left to us. | :*We return it back to the rank of the hundreds; so, they are 10. We divide them by 3; the quotient is 3, 1 is left to us. | ||
| − | |style="text-align:right;"|נשיבהו | + | |style="text-align:right;"|נשיבהו אחורנית במעלת המאות‫<ref group=note>P1051 marg. פי' נחשוב אותו עשרה</ref> והיו י' נחלקנו על ג' ועלה בחלוק ג' נשאר לנו א‫'‫<ref group=II>נשיבהו: V397 נשיבהו נשיבהו<br> |
| + | במעלת: Mo30 ונכתבנו במעלת; V397; V398 במעלה<br> | ||
| + | והיו: Mo30 ויהיו; V397 היו; V398 והוא<br> | ||
| + | והיו י‫': P1051 om.<br> | ||
| + | נחלקנו: Mo30 ואותם העשרה נחלק<br> | ||
| + | בחלוק: V397 בחלק<br>ויעלה בחלוק: Mo30 ויצאו<br> | ||
| + | נשאר: Mo30 ונשאר<br> | ||
| + | א‫': Mo30 א' במקום המאות</ref> | ||
|- | |- | ||
| | | | ||
:*We return it back to the rank of the tens; so, they are 10. We add them to what is written in the second rank; they are 13. We divide them by 3 and give it 4; so, we are left with 1. | :*We return it back to the rank of the tens; so, they are 10. We add them to what is written in the second rank; they are 13. We divide them by 3 and give it 4; so, we are left with 1. | ||
| − | |style="text-align:right;"|השבנוהו<ref group=II>השבנוהו: Mo30 ונשיבהו; P1051; V398 השיבנו אותו< | + | |style="text-align:right;"|השבנוהו אחורנית במעלת העשרות ויהיו י' חברנו אותו עם הכתוב במעלה השנית היו י"ג חלקנו אותו על ג' ונתנו לו ד' נשאר לנו א‫'‫<ref group=II>השבנוהו: Mo30 ונשיבהו; P1051; V398 השיבנו אותו<br> |
| + | ויהיו: V397 היה<br> | ||
| + | במעלה: P1051 למעלה<br> | ||
| + | ויהיו ... השנית: V398 om.<br> | ||
| + | במעלת העשרות ... במעלה השנית: Mo30 ונחבר אליו הג' אשר בעשרות<br> | ||
| + | היו: Mo30 ויהיו<br> | ||
| + | י"ג: V398 י"ג עם הג' שעל המעלה ההיא<br> | ||
| + | חלקנו אותו: Mo30 נחלקם; V398 חלק<br> | ||
| + | ונתנו: P1051; V397 נתנו<br> | ||
| + | לו: P1051; V397 להם<br> | ||
| + | ונתנו לו: Mo30 ויצאו<br> | ||
| + | ונתנו לו ד‫': V398 יהיו ד‫'<br> | ||
| + | נשאר: Mo30 ונשאר; P1051 נשארו<br> | ||
| + | לנו: Mo30 om.<br> | ||
| + | א‫': Mo30 אחד במקום העשרה והוא פחות מהמספר החולק</ref> | ||
|- | |- | ||
| | | | ||
:*We return it back to the first rank; they are 12 that cannot be divided, because the remainder is less than the divisor, and everything is already taken out. | :*We return it back to the first rank; they are 12 that cannot be divided, because the remainder is less than the divisor, and everything is already taken out. | ||
| − | |style="text-align:right;"|השיבונוהו<ref group=II>השיבונוהו: Mo30 ונשיבם; P1051 השיבונו אותו< | + | |style="text-align:right;"|השיבונוהו אחורנית במעלה הראשונה היו י"ב שלא יתחלקו כי הנשאר פחות מאותו המחולק עליו וכבר יצא לחוץ‫<ref group=II>השיבונוהו: Mo30 ונשיבם; P1051 השיבונו אותו<br> |
| + | נשאר לנו א' השיבונוהו אחורנית: V398 כתוב; marg. ד' במעלה הראשונה והנה נשאר לנו אחד תשיבהו אחורנית<br> | ||
| + | במעלה הראשונה: Mo30 ר"ל אל האחדים ועם הב' אשר באחדים<br> | ||
| + | היו: Mo30 יהיו; P1051; V398 והיו<br> | ||
| + | י"ב: V398 י"ב marg. עם ב' שעל המעלה הר' שלא‫'<br> | ||
| + | שלא: Mo30 ולא<br> | ||
| + | הנשאר: Mo30 הם<br> | ||
| + | מאותו: Mo30 מהמספר<br> | ||
| + | המחולק: V397 המחובר<br> | ||
| + | וכבר: P1051 וככה</ref> | ||
|- | |- | ||
|If we want to divide one digit or two digits or many digits by one digit or two digits or three or many, provided that they are less than the digits in the top row, you proceed as follows: | |If we want to divide one digit or two digits or many digits by one digit or two digits or three or many, provided that they are less than the digits in the top row, you proceed as follows: | ||
| − | |style="text-align:right;"|וכאשר נרצה לחלק מספר אחד או שני<ref group=II>שני: Mo30 שנים< | + | |style="text-align:right;"|וכאשר נרצה לחלק מספר אחד או שני מספרים או מספרים רבים על מספר אחד או על שני מספרים או על שלשה או על רבים על מנת שיהיו פחותים ממספרי הטור העליון ככה תעשה‫<ref group=II>שני: Mo30 שנים<br> |
| + | מספרים: P1051 <s>ג‫'</s> מספרים<br> | ||
| + | שני: P1051 om.<br> | ||
| + | שני מספרים: V397 שנים<br> | ||
| + | על: Mo30 om.<br> | ||
| + | על: Mo30; V398 om.<br> | ||
| + | או על שלשה או על: P1051 om.</ref> | ||
| + | |- | ||
| + | |Give the last in the bottom row of the top row, as much as you can give it from the last digit in the top row. | ||
| + | |style="text-align:right;"|<div id="give_the_last_in_the_bottom_row_of_the_top_row"></div>תן לאחרון שבטור השפל מן הטור העליון מה שתוכל לתת לו מהמספר האחרון שבטור העליון‫<ref group=note>[[פירוש_אנונימי_לספר_המספר#Give_the_last_in_the_bottom_row_of_the_top_row|<span style=color:blue>commentary: give the last in the bottom row of the top row</span>]]</ref>‫<ref group=II>מן הטור: Mo30; P1051; V398 מהטור<br> | ||
| + | מהמספר: V398 מן המספר</ref> | ||
|- | |- | ||
| − | |Give | + | |Give the preceding in the bottom row, which is first to the last, as much as the product of the number you gave the last multiplied by the digit in the bottom row that precedes the last digit. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<div id="give_the_preceding_in_the_bottom_row"></div>ותן לראשון מן הטור השפל שהוא ראשון לאחרון<ref group=note>ראשון לאחרון: Mo30 marg. ר"ל קודם האחרון</ref> ככפל המספר שנתת לאחרון על מספר הטור השפל שהוא לפני האחרון‫<ref group=note>[[פירוש_אנונימי_לספר_המספר#Give_the_preceding_in_the_bottom_row|<span style=color:blue>commentary: give the preceding in the bottom row</span>]]</ref>‫<ref group=II>ותן: Mo30; P1051; V397 ותתן<br> |
| + | מן הטור: Mo30 מהטור<br> | ||
| + | על מספר: V398 <s>על</s> <sup>מ</sup>מספר</ref> | ||
|- | |- | ||
|If you cannot do this, reduce the number that you gave it first. | |If you cannot do this, reduce the number that you gave it first. | ||
| − | |style="text-align:right;"|ואם לא תוכל לעשות<ref group= | + | |style="text-align:right;"|<div id="If_you_cannot_do_this"></div>ואם לא תוכל לעשות ככה שוב וגרע ממספרך שנתת לו בתחלה‫<ref group=note>[[פירוש_אנונימי_לספר_המספר#If_you_cannot_do_this|<span style=color:blue>commentary: if you cannot do this</span>]]</ref>‫<ref group=II>תוכל לעשות: V397 illegible<br> |
| + | ממספרך: P1051 עד ע' ממספרך</ref> | ||
|- | |- | ||
|When you have to take any digit from the digit that precedes the last, return it back as tens. | |When you have to take any digit from the digit that precedes the last, return it back as tens. | ||
| − | |style="text-align:right;"|וכשאתה צריך לקחת מהטור<ref group=II>מהטור: P1051 top</ref> | + | |style="text-align:right;"|<div id="when_you_have_to_take_any_digit_from_the_digit_that_precedes_the last"></div>וכשאתה צריך לקחת מהטור שהוא לפני האחרון שום מספר השיבהו אחורנית לעשרות‫<ref group=note>[[פירוש_אנונימי_לספר_המספר#When_you_have_to_take_any_digit_from_the_digit_that_precedes_the_last|<span style=color:blue>commentary: when you have to take any digit from the digit that precedes the last</span>]]</ref>‫<ref group=II>מהטור: P1051 top</ref> |
|- | |- | ||
|This is how you proceed with all the ranks. | |This is how you proceed with all the ranks. | ||
| Line 1,283: | Line 2,446: | ||
|- | |- | ||
|If there is a zero in one of the ranks of the upper row, return back from the higher rank next to it as tens and take from them as much as is necessary. | |If there is a zero in one of the ranks of the upper row, return back from the higher rank next to it as tens and take from them as much as is necessary. | ||
| − | |style="text-align:right;"|ואם<ref group= | + | |style="text-align:right;"|ואם היה באחת ממעלות הטור העליון גלגל <div id="return_back_from_the_higher_rank"></div>השב מן הגבוה ממנו אחורנית בעשרות וקח ממנו מה שצריך לו‫<ref group=note>[[פירוש_אנונימי_לספר_המספר#Return_back_from_the_higher_rank|<span style=color:blue>commentary: return back from the higher rank</span>]]</ref>‫<ref group=II>ואם: V398 אם<br> |
| + | ממעלות: V397 מהמעלות<br> | ||
| + | מן הגבוה: V397 מהגבוה<br> | ||
| + | אחורנית: V398 om.<br> | ||
| + | ממנו: V397 מהם</ref> | ||
|- | |- | ||
|If there are two zeros in the ranks of the upper row and in the ranks of the lower row there are numbers, you return back the higher [number] that corresponds to the digit that follows the last zero and take what you need from it. Then you return the remainder back as tens, and take what you need for the multiple of the number that is in the bottom row from the rank from which it should be taken. | |If there are two zeros in the ranks of the upper row and in the ranks of the lower row there are numbers, you return back the higher [number] that corresponds to the digit that follows the last zero and take what you need from it. Then you return the remainder back as tens, and take what you need for the multiple of the number that is in the bottom row from the rank from which it should be taken. | ||
| − | |style="text-align:right;"|ואם היו שני<ref group=II>שני: Mo30; P1051; V398 שנים< | + | |style="text-align:right;"|ואם היו שני גלגלים במעלות הטור העליון ובמעלות הטור השפל מספרים תשיב אחורנית הגבוה שהוא כנגד החשבון שהוא אחר הגלגל האחרון ותקח מהם מה שתצטרך ומהנשאר תשיב אחורנית בעשרות ותקח ממנו מה שתצטרך בכפל המספר שהוא בטור השפל מן המעלה שהיא ראויה לקחת ממנה‫<ref group=II>שני: Mo30; P1051; V398 שנים<br> |
| + | במעלות: Mo30 למעלות; P1051; V397 במעלת<br> | ||
| + | ובמעלות: Mo30; V397 ובמעלת<br> | ||
| + | מספרים: V397 om.<br> | ||
| + | תשיב: Mo30 השב<br> | ||
| + | אחר: Mo30 אחרון<br> | ||
| + | האחרון: V397 om.<br> | ||
| + | מהם: V398 <s>מהם</s> <sup>ממנו</sup><br> | ||
| + | שתצטרך: Mo30 שצריך; V397 שיצטרך<br> | ||
| + | ומהנשאר: Mo30 ומה שנשאר; P1051 ומהשאר<br> | ||
| + | ומהנשאר ... שתצטרך: V397 marg.<br> | ||
| + | בכפל: V397 בכל<br> | ||
| + | שהוא: V397 שהוא אחר הגלגל ותקח מהם<br> | ||
| + | מן המעלה: V397 מהמעלה<br> | ||
| + | ממנה: V397 ממנו</ref> | ||
|- | |- | ||
| | | | ||
*{{#annot:8213÷353|157|ZaHA}}Example: we wish to divide 8213 by 353. | *{{#annot:8213÷353|157|ZaHA}}Example: we wish to divide 8213 by 353. | ||
:<math>\scriptstyle8213\div353</math> | :<math>\scriptstyle8213\div353</math> | ||
| − | |style="text-align:right;"|<big>דמיון זה</big>‫<ref group=II>זה: Mo30 כזו הצורה marg. רצינו לחלק ח' אלפים ורי"ג על שנ"ג; P1051; V397 וזה; V398 om.</ref> | + | |style="text-align:right;"|<big>דמיון זה</big>{{#annotend:ZaHA}}‫<ref group=II>זה: Mo30 כזו הצורה marg. רצינו לחלק ח' אלפים ורי"ג על שנ"ג; P1051; V397 וזה; V398 om.</ref> |
|- | |- | ||
| | | | ||
| Line 1,355: | Line 2,536: | ||
| | | | ||
:*The 94 that we wrote is the remainder of the number that cannot be divided. | :*The 94 that we wrote is the remainder of the number that cannot be divided. | ||
| − | |style="text-align:right;"|ד'ט'<ref group=II> | + | |style="text-align:right;"|ד'ט' שכתבנו הוא הנשאר מן החשבון <ref group=II>MS St.P569 excerpt 2 begin.</ref>שלא יתחלק‫<ref group=II>ד'ט‫': P1051 om.<br> |
| + | הוא: V397 והוא<br> | ||
| + | הנשאר: V397 הנשמר<br> | ||
| + | מן החשבון: P1051; V397 מחשבון<br> | ||
| + | יתחלק: P1051 נתחלק כזאת הצורה; V397 נתחלק<br> | ||
| + | ד'ט' ... יתחלק: Mo30; V398 om.</ref> | ||
|- | |- | ||
| | | | ||
:*When we divide 8, which is the last [digit] of the upper row, by 3, which is the last [digit] of the lower row, we give it two, and because the 3 of the lower row is third, we return it back to the third [digit] from there, and it reaches the rank of tens. We are left with two on the digit 8. | :*When we divide 8, which is the last [digit] of the upper row, by 3, which is the last [digit] of the lower row, we give it two, and because the 3 of the lower row is third, we return it back to the third [digit] from there, and it reaches the rank of tens. We are left with two on the digit 8. | ||
| − | |style="text-align:right;"|והנה<ref group=II>והנה: Mo30 הנה< | + | |style="text-align:right;"|והנה כאשר חלקנו ח' שהוא אחרון בטור העליון על ג' שהוא אחרון בטור השפל והנה נתנו לו שנים ובעבור שהיה הג' שבטור השפל שלישי החזרנו לשלישי ממנו אחורנית והגיע למעלת העשרות ונשאר לנו במספר הח' שנים‫<ref group=II>והנה: Mo30 הנה<br> |
| + | והנה כאשר: V398 <s>וכאשר</s> והנה כאשר<br> | ||
| + | שהוא: Mo30 <sup>ש</sup><s>ש</s>הוא<br> | ||
| + | על: Mo30 עם<br> | ||
| + | אחרון: V397 האחרון<br> | ||
| + | נתנו: Mo30 נתן<br> | ||
| + | שהיה: P1051; V397 כי היה<br> | ||
| + | הג': Mo30 בג‫'<br> | ||
| + | שלישי: Mo30 במעלה השלישית<br> | ||
| + | החזרנו: P1051 החזירהו<br> | ||
| + | לשלישי: Mo30 לשלישית<br> | ||
| + | למעלת: P1051 למעלות<br> | ||
| + | הח‫': P1051 ח‫'</ref> | ||
|- | |- | ||
| | | | ||
:*We leave there one, because one is enough for us, and return the one back to the two; they are 12. We subtract from them 10, which is double five, which is the middle in the bottom row, so 2 are left on the 2. | :*We leave there one, because one is enough for us, and return the one back to the two; they are 12. We subtract from them 10, which is double five, which is the middle in the bottom row, so 2 are left on the 2. | ||
| − | |style="text-align:right;"|והנה הנחנו שם אחד<ref group=II>אחד: V398 top< | + | |style="text-align:right;"|והנה הנחנו שם אחד כי אחד יספיק לנו והחזרנו האחד אצל השנים והיו י"ב וחסרנו ממנו י' שהוא כפל חמשה האמצעי שבטור השפל והנה נשארו ב' על הב‫'‫<ref group=II>אחד: V398 top<br> |
| + | האחד: Mo30 הא' לאחור<br> | ||
| + | והיו: Mo30 והוא; P1051 יהיו<br> | ||
| + | וחסרנו: P1051 חסרנו<br> | ||
| + | הב‫': V397 ב‫'</ref> | ||
|- | |- | ||
| | | | ||
:*We leave there one and return one back on the one, which is third; they are 11. We subtract from them 6 for 3, which is first in the bottom row; so, 5 remain. | :*We leave there one and return one back on the one, which is third; they are 11. We subtract from them 6 for 3, which is first in the bottom row; so, 5 remain. | ||
| − | |style="text-align:right;"|נניח שם אחד ונחזיר אחד אחורנית על אחד שהוא שלישי ויהיו | + | |style="text-align:right;"|נניח שם אחד ונחזיר אחד אחורנית על אחד שהוא שלישי ויהיו י"א נסיר ממנו ו' לג' שהוא ראשון שבטור השפל נשארו ה‫'‫<ref group=II>ויהיו: Mo30 יהיו<br> |
| + | ו‫': V398 ב‫' marg. ב' פעמי' שהוא ו‫'<br> | ||
| + | שבטור: P1051 לטור<br> | ||
| + | ממנו ו' ... שבטור השפל: Mo30 לג' שהוא ראשון שבטור השפל כפל ב' פעמים ג' והם ו' והסירם<br> | ||
| + | נשארו ה‫': V397 illegible</ref> | ||
|- | |- | ||
| | | | ||
:Thus, all 3 lower [digits] have what they are entitled to. | :Thus, all 3 lower [digits] have what they are entitled to. | ||
| − | |style="text-align:right;"|והנה<ref group=II>והנה: Mo30 והנה נתת; P1051 <s>ת</s> הנה; V398 הנה</ref> | + | |style="text-align:right;"|והנה לכל הג' השפלים מה שראוי להם‫<ref group=II>והנה: Mo30 והנה נתת; P1051 <s>ת</s> הנה; V398 הנה</ref> |
|- | |- | ||
| | | | ||
:We divide again, because are left with 1 on the 8, 1 on the 2 and 5 on the 1. | :We divide again, because are left with 1 on the 8, 1 on the 2 and 5 on the 1. | ||
| − | |style="text-align:right;"|נשוב<ref group=II>נשוב: V398 נשיב< | + | |style="text-align:right;"|נשוב לחלק כי נשאר לנו א' על ח' וא' על ב' וה' על א‫'‫<ref group=II>נשוב: V398 נשיב<br> |
| + | נשאר: Mo30 נשארו; V397 illegible</ref> | ||
|- | |- | ||
| colspan=2| | | colspan=2| | ||
| Line 1,399: | Line 2,606: | ||
| | | | ||
:*We return the 1, which is on the 8 back on the 1 above the 2; they are 11. We divide them by the 3, which is in the bottom row; they are 3. We write it corresponding to the first digit, which precedes the two; so, we are left with 2. | :*We return the 1, which is on the 8 back on the 1 above the 2; they are 11. We divide them by the 3, which is in the bottom row; they are 3. We write it corresponding to the first digit, which precedes the two; so, we are left with 2. | ||
| − | |style="text-align:right;"|נשיב הא' שהוא<ref group=II>שהוא: Mo30 om.<br>הא' שהוא: V397 om.< | + | |style="text-align:right;"|נשיב הא' שהוא על הח' אחורנית על א' אשר על הב' יהיו י"א נחלק אותם על ג' שהוא בטור השפל יהיו ג' ונכתבנו כנגד הטור הראשון שהוא לפני השנים ונשארו לנו ב‫'‫<ref group=II>שהוא: Mo30 om.<br> |
| + | הא' שהוא: V397 om.<br> | ||
| + | הח‫': Mo30 הא‫'; V398 ח‫'<br> | ||
| + | על א' אשר על הב‫': Mo30; P1051; V397 om.<br> | ||
| + | יהיו: Mo30 ויהיו<br> | ||
| + | שהוא: P1051 om.<br> | ||
| + | שהוא בטור: Mo30 שבטור<br> | ||
| + | שהוא בטור השפל יהיו ג‫': V397 om.<br> | ||
| + | ונכתבנו: Mo30 וכתבנו<br> | ||
| + | השנים: V397 ב‫'<br> | ||
| + | ונשארו: Mo30 נשארו</ref> | ||
|- | |- | ||
| | | | ||
:*We return them back on the 5; they are 25. We give 15 to the 5 that is middle in the lower row; 10 remain. | :*We return them back on the 5; they are 25. We give 15 to the 5 that is middle in the lower row; 10 remain. | ||
| − | |style="text-align:right;"|נשיבם על הה'<ref group=II>על הה': P1051; V397 om.< | + | |style="text-align:right;"|נשיבם על הה' אחורנית יהיו כ"ה נתן לחמשה האמצעי שבטור השפל ט"ו ונשארו עשרה‫<ref group=II>על הה‫': P1051; V397 om.<br> |
| + | על הה' אחורנית: Mo30 אחורנית על הה‫'<br> | ||
| + | האמצעי: Mo30 האמצעית; P1051 האמצעים<br> | ||
| + | נתן ... השפל: V398 נסיר<br> | ||
| + | ונשארו: Mo30; P1051 נשארו; V397 ונשארו לנו</ref> | ||
|- | |- | ||
| | | | ||
:*We return one back on the 3; 9 remain. The 1 with the 3 are 13; we take from them 9 and 4 remain. | :*We return one back on the 3; 9 remain. The 1 with the 3 are 13; we take from them 9 and 4 remain. | ||
| − | |style="text-align:right;"|נשיב אחד אחורנית על הג' | + | |style="text-align:right;"|נשיב אחד אחורנית על הג‫'‫<ref group=note>P1051 marg. פי' ק' כדי שיתחלק עליהם ג' ראשון שבטור השפל ויהיו י"ג נחלקם על הג' נתן לו ג' ואע"פ שיספיק לתת ד' לא נתן עולה כי אם בשוה מה שנתננו לאחדים בחלוק וישארו ד' על הג‫'</ref> וישארו ט' והא' עם הג' י"ג ונקח מהם ט' וישארו ד‫'‫<ref group=II>הג‫': Mo30 ג‫'<br> |
| + | הג‫': Mo30 ג‫'<br> | ||
| + | י"ג: Mo30 יהיו י"ג<br> | ||
| + | ונקח: P1051 נקח; V397 ויקח<br> | ||
| + | מהם: Mo30 ממנו<br> | ||
| + | ט‫': Mo30 ט' מי"ג<br> | ||
| + | וישארו: P1051; V397 ישארו<br> | ||
| + | ד‫': Mo30 ד' על ג‫'</ref> | ||
|- | |- | ||
| | | | ||
:*The 9 on the 5, because we cannot take the 3 out of the 10 first, although it was enough for it, for it is not the same rank now; although it was taken first, it was first third to it, since the last of the bottom row took from the 8, but now it took from the 2, and since it is third, it must take from the same rank. So, the remainder is 94. | :*The 9 on the 5, because we cannot take the 3 out of the 10 first, although it was enough for it, for it is not the same rank now; although it was taken first, it was first third to it, since the last of the bottom row took from the 8, but now it took from the 2, and since it is third, it must take from the same rank. So, the remainder is 94. | ||
| − | |style="text-align:right;"|וט' על הה'<ref group=II> | + | |style="text-align:right;"|וט' על הה' כי לא יכולנו לקחת בראשונה הג' מהי' אע"פ שיספיק לו כי אינו מעלתו עכשיו אע"פ שלקח ממנו בראשונה כי בראשונה היה שלישי לו כי האחרון שבטור השפל לקח מן הח' אבל עתה לקח מן הב' א' ואחר שהוא שלישי צריך הוא שיקח ממעלתו השלישית<ref group=II>MS St.P569 excerpt 2 end</ref> והנה הנשאר ד' ט‫'‫<ref group=II>הה‫': P1051 האחד<br> |
| + | יכולנו: Mo30 יוכלו; V397 יכול<br> | ||
| + | בראשונה: P1051 כבראשונה; V398 עתה marg. <s>בראשונה</s><br> | ||
| + | מהי‫': Mo30 מהט‫'<br> | ||
| + | לו: Mo30 לנו<br> | ||
| + | מעלתו עכשיו: Mo30 עתה מעלתו<br> | ||
| + | הג' מהי' ... ממנו בראשונה: P1051 om.<br> | ||
| + | בראשונה: V398 הראשונה<br> | ||
| + | היה: V397 היו<br> | ||
| + | שלישי: Mo30 שלישית<br> | ||
| + | הח' אבל עתה לקח מן: Mo30 om.<br> | ||
| + | הב‫': Mo30 ב‫'<br> | ||
| + | א‫': Mo30; P1051; V398 om.<br> | ||
| + | הוא: Mo30; P1051; V397 om.<br> | ||
| + | שיקח: Mo30; V398 שנקח<br> | ||
| + | ממעלתו: Mo30 מן מעלתו<br> | ||
| + | השלישית: Mo30 הג' השלישי; P1051; V398 השלישי<br> | ||
| + | והנה: Mo30 והיה; V397 הנה<br> | ||
| + | הנשאר: Mo30 הנשאר בלתי מחולק בט‫'; V397 נשאר<br> | ||
| + | ד'ט‫': Mo30 marg. והנשאר ד'ט‫'; P1051; V398 ט'ד‫'</ref> | ||
|- | |- | ||
| | | | ||
*{{#annot:9381÷296|157|05kI}}Another example. | *{{#annot:9381÷296|157|05kI}}Another example. | ||
:<math>\scriptstyle9381\div296</math> | :<math>\scriptstyle9381\div296</math> | ||
| − | |style="text-align:right;"|<big>דמיון אחר</big><ref group=II>אחר: P1051 <s>ב</s>אחר</ref> | + | |style="text-align:right;"|<big>דמיון אחר</big>{{#annotend:05kI}}‫<ref group=II>אחר: P1051 <s>ב</s>אחר</ref> |
|- | |- | ||
| | | | ||
| Line 1,480: | Line 2,727: | ||
| | | | ||
:*We want to divide 9 by two, but we cannot give it 4, because then only 1 remains, and when you return it back, with the 3 they are 13, but 4 times 9 is 36. So, we give it 3; 3 remain. | :*We want to divide 9 by two, but we cannot give it 4, because then only 1 remains, and when you return it back, with the 3 they are 13, but 4 times 9 is 36. So, we give it 3; 3 remain. | ||
| − | |style="text-align:right;"|באנו<ref group=II>באנו: V397 בקשנו< | + | |style="text-align:right;"|באנו לחלק ט' על שנים והנה לא יכולנו לתת לו ד' כי לא ישאר אלא א' וכאשר תשיבהו אחורנית הנה עם הג' י"ג וט' ד' פעמים ל"ו על כן נתן לו ג' נשארו ג‫'‫<ref group=II>באנו: V397 בקשנו<br> |
| + | לחלק: Mo30 לחלק מספר כמו שהוא לפניך הנה אנו צריכין לחלק<br> | ||
| + | ט‫': Mo30 ט' אחרונה שבטור העליון<br> | ||
| + | שנים: Mo30 ב' אחרונה שבטור השפל<br> | ||
| + | יכולנו: V397 יכול<br> | ||
| + | ישאר: Mo30 נשאר<br> | ||
| + | תשיבהו: V397 נשיבהו<br> | ||
| + | הנה: Mo30 הנה עלו; V397 om.<br> | ||
| + | עם הג‫': Mo30 om.<br> | ||
| + | ל"ו: Mo30 עלו ל"ו<br> | ||
| + | על כן: P1051; V397 om.<br> | ||
| + | נשארו: Mo30 ונשארו</ref> | ||
|- | |- | ||
| | | | ||
:*We return all back to the second to it, which is 3; they are 33 there. We give 3 to the 9; they are 27; 6 remain on the 3. | :*We return all back to the second to it, which is 3; they are 33 there. We give 3 to the 9; they are 27; 6 remain on the 3. | ||
| − | |style="text-align:right;"|נשיבם כלם<ref group=II>נשיבם כלם: Mo30 כלם נשיבם< | + | |style="text-align:right;"|נשיבם כלם אחורנית לשני לו שהוא ג' ויהיו שם ל"ג נתן לט' ג' יהיו כ"ז נשארו ו' על הג‫'‫<ref group=II>נשיבם כלם: Mo30 כלם נשיבם<br> |
| + | ויהיו: P1051 יהיו<br> | ||
| + | ויהיו שם: V397 ...הוא<br> | ||
| + | לט‫': V398 לו לט‫'<br> | ||
| + | יהיו: Mo30 ויהיו; P1051 יהיה<br> | ||
| + | נשארו: Mo30 ונשארו<br> | ||
| + | הג‫': Mo30 ג‫'</ref> | ||
|- | |- | ||
| | | | ||
:*We take one from them and leave 5. We return it back on the 8, which is the third from the last of the top row; with the 8 they are 18. We give all to the 6, which is the third of the bottom row. We write a zero on the 8, because there is nothing left on the 8. | :*We take one from them and leave 5. We return it back on the 8, which is the third from the last of the top row; with the 8 they are 18. We give all to the 6, which is the third of the bottom row. We write a zero on the 8, because there is nothing left on the 8. | ||
| − | |style="text-align:right;"|נקח<ref group=II>נקח: Mo30 ונקח< | + | |style="text-align:right;"|נקח מהם א' ונניח ה' נשיבהו על הח' שהוא שלישי לאחרון שבטור העליון ועם הח' יהיו י"ח נתן אותם כלם לו' שהוא שלישי שבטור השפל נכתוב על הח' גלגל לפי שלא נשאר על הח' מאומה‫<ref group=II>נקח: Mo30 ונקח<br> |
| + | מהם א‫': Mo30 <sup>א‫'</sup> מהם<br> | ||
| + | נשיבהו: P1051 נשימהו; V397 נשימהו נשימהו<br> | ||
| + | שלישי: Mo30 שלישית<br> | ||
| + | לאחרון: Mo30; P1051; V398 לאחריו<br> | ||
| + | יהיו: V397 שהוא; Mo30; V398 הוא<br> | ||
| + | נתן: Mo30 ונתן<br> | ||
| + | אותם: V398 לו<br> | ||
| + | נכתוב: Mo30 ונכתוב<br> | ||
| + | נשאר: Mo30 נשאר ממנו<br> | ||
| + | על הח‫': Mo30 om.</ref> | ||
|- | |- | ||
| colspan=2| | | colspan=2| | ||
| Line 1,512: | Line 2,786: | ||
| | | | ||
:*We divide again, because not everything has been taken out yet. We take two, from the 5 that we left on the 3, which is the second in the top row; [the multiple] is one. We write this one on the 6 after the 3, which we put on the 9 in the first division, which is the third in the bottom row, then everything has already been removed. | :*We divide again, because not everything has been taken out yet. We take two, from the 5 that we left on the 3, which is the second in the top row; [the multiple] is one. We write this one on the 6 after the 3, which we put on the 9 in the first division, which is the third in the bottom row, then everything has already been removed. | ||
| − | |style="text-align:right;"|נשוב לחלק שעדין | + | |style="text-align:right;"|נשוב לחלק שעדין לא יצא לחוץ נקח מן הה' שהנחנו על הג' שהוא שני בטור העליון שנים שהוא אחד נכתוב זה האחד על הו' אחרי הג' ששמנו על הט' בחלוק הראשון והוא שלישי בטור השפל וכבר יצא לחוץ‫<ref group=II>שעדין: P1051 <sup>ש</sup>עדין<br> |
| + | שהוא: Mo30 בטור שהם; P1051; V397 שהם; V398 שהם marg. שהוא<br> | ||
| + | נכתוב: V397 ונכתוב<br> | ||
| + | זה האחד: Mo30 האחד הזה<br> | ||
| + | אחרי הג' ששמנו על הט‫': P1051 om.<br> | ||
| + | הראשון: P1051 ראשון<br> | ||
| + | שלישי: Mo30 <s>ג‫'</s> שלישי</ref> | ||
|- | |- | ||
| | | | ||
:*We take one from the 3 that is above the 3; two remain. We give the one to the zero; they are 10. We give 9 to the 9 that is in the bottom row; 1 remains on the zero. | :*We take one from the 3 that is above the 3; two remain. We give the one to the zero; they are 10. We give 9 to the 9 that is in the bottom row; 1 remains on the zero. | ||
| − | |style="text-align:right;"|נקח מן הג' | + | |style="text-align:right;"|נקח מן הג' שעל הג' אחד וישארו שנים נתן האחד על הגלגל והם י' נתן לט' שבטור השפל ט' נשאר על הגלגל א‫'‫<ref group=II>מן הג‫': Mo30; V397 מהג‫'<br> |
| + | שבטור: V398 שהוא בטור</ref> | ||
|- | |- | ||
| | | | ||
:*We return it back on the 1, which is the fourth foremost in the top row; they are 11. We give it 6; 5 remain on the 1. | :*We return it back on the 1, which is the fourth foremost in the top row; they are 11. We give it 6; 5 remain on the 1. | ||
| − | |style="text-align:right;"|נשיבהו אחורנית על הא' שהוא רביעי וראשון | + | |style="text-align:right;"|נשיבהו אחורנית על הא' שהוא רביעי וראשון בטור העליון והם י"א נתן לו ו' נשארו ה' על הא‫'‫<ref group=II>וראשון: P1051 והראשון<br> |
| + | נשארו: Mo30 ונשארו</ref> | ||
|- | |- | ||
| | | | ||
:There remainders on the top row are: 5, zero and 2, which are 205. These cannot be divided further, because the 3 [digits] in the bottom row are 296. So, the taken for each [= the quotient] is 31. | :There remainders on the top row are: 5, zero and 2, which are 205. These cannot be divided further, because the 3 [digits] in the bottom row are 296. So, the taken for each [= the quotient] is 31. | ||
| − | |style="text-align:right;"|הנה | + | |style="text-align:right;"|הנה הנשארים על הטור העליון ה' וגלגל וב' שהם ר"ה ולא יתחלקו יותר שהג' שבטור השפל הם רצ"ו והנלקח לכל אחד ל"א‫<ref group=II>הנה: Mo30 והנה<br> |
| + | הנשארים: Mo30 נשארו<br> | ||
| + | ר"ה: Mo30 <s>ר"א</s> <sup>ר"ה</sup><br> | ||
| + | יותר: V397 נותר<br> | ||
| + | שבטור: V397 שהם בתור<br> | ||
| + | ולא ... רצ"ו: Mo30; P1051; V398 om.</ref> | ||
|- | |- | ||
| | | | ||
| Line 1,605: | Line 2,892: | ||
| | | | ||
:*We want to divide the top row by the bottom row. We cannot give it 2, because then only 1 remains and with 4 they are 14, but we have to divide it by 9 twice. So, we only give it 1 and write it corresponding to the 9 that is in the top row, which is fourth to the 5 back, as the two of the lower row, which is fourth to the first 5 in the bottom row. 3 remain on the 5. | :*We want to divide the top row by the bottom row. We cannot give it 2, because then only 1 remains and with 4 they are 14, but we have to divide it by 9 twice. So, we only give it 1 and write it corresponding to the 9 that is in the top row, which is fourth to the 5 back, as the two of the lower row, which is fourth to the first 5 in the bottom row. 3 remain on the 5. | ||
| − | |style="text-align:right;"|בקשנו לחלק הטור העליון על הטור השפל<ref group= | + | |style="text-align:right;"|בקשנו לחלק הטור העליון על הטור השפל לתת לו<ref group=note>לו: P1051 marg. פי' לאות האחרון מהטור העליון</ref> ב' לא נוכל שלא ישאר כי אם א' וד' והם י"ד ויש לנו לחלק על ט'<ref group=note>P1051 marg פי' ק' ולא יספיק לתת ב' לט' כאשר נתננו לב'</ref> ב' פעמים אך נתן לו א' ונשים אותו כנגד ט' שהם בטור העליון שהוא רביעי לה' אחורנית כשנים בטור השפל שהוא רביעי לה' ראש שבטור השפל נשארו ג' על הה‫'‫<ref group=II>השפל: P1051 השפל כמו שכתוב ומצוייר לפניך בזאת הצורה marg. והנה<br> |
| + | ב‫': P1051 ב' פעמים <sup>ב‫'</sup><br> | ||
| + | לא: V397 ולא<br> | ||
| + | לא נוכל: Mo30; V398 אי אפשר<br> | ||
| + | שלא: Mo30 כי לא<br> | ||
| + | אם: V398 top<br> | ||
| + | וד‫': V397 ועם ד‫'<br> | ||
| + | והם: V397 om.; Mo30; V398 הם<br> | ||
| + | והם י"ד: P1051 om.<br> | ||
| + | ויש לנו: Mo30 וישארו<br> | ||
| + | ב' פעמים: Mo30; P1051; V397; V398 om.<br> | ||
| + | אך נתן: V398 ונתן<br> | ||
| + | אך נתן לו: Mo30 ולכן לא נתן רק<br> | ||
| + | ונשים אותו: V397 om.<br> | ||
| + | ט‫': Mo30; P1051 הט‫'<br> | ||
| + | שהם: Mo30 om.<br> | ||
| + | כשנים: P1051 om.; V398 כמו הב‫'<br> | ||
| + | בטור: P1051 שבטור; V397 שהוא בטור<br> | ||
| + | השפל: P1051 העליון<br> | ||
| + | שהוא: P1051 כי ב' שבטור השפל הוא<br> | ||
| + | ראש: P1051 ראשונה<br> | ||
| + | שבטור: V397 שהוא בטור<br> | ||
| + | על: V397 והם ג' על<br> | ||
| + | הה‫': Mo30 ה‫'</ref> | ||
|- | |- | ||
| | | | ||
:*We take one from them; 2 remain on the 5. We return it back on the 4; they are 14. 9 are taken; 5 remain. | :*We take one from them; 2 remain on the 5. We return it back on the 4; they are 14. 9 are taken; 5 remain. | ||
| − | |style="text-align:right;"|נקח מהם אחד<ref group=II>מהם אחד: V398 אחד מהם< | + | |style="text-align:right;"|נקח מהם אחד ישארו ב' על הה' נשיבהו אחורנית על הד' יהיו י"ד יקח ט' ישארו ה‫'‫<ref group=II>מהם אחד: V398 אחד מהם<br> |
| + | ישארו: Mo30 נשארו<br> | ||
| + | ישארו ב' על הה‫': V398 om.<br> | ||
| + | נשיבהו: Mo30 ונשיבהו<br> | ||
| + | יהיו י"ד: Mo30; V397; V398 om.<br> | ||
| + | יקח: Mo30 נקח<br> | ||
| + | יקח ט‫': V397 om.</ref> | ||
|- | |- | ||
| | | | ||
:*The 4 in the bottom row must take from the third in the top row, because it is third, but we cannot, because the third in the top row is a zero. So, we return one from the 5 that we left on the 4 back on the zero; they are 10. 4 are taken; 6 remain on the zero. | :*The 4 in the bottom row must take from the third in the top row, because it is third, but we cannot, because the third in the top row is a zero. So, we return one from the 5 that we left on the 4 back on the zero; they are 10. 4 are taken; 6 remain on the zero. | ||
| − | |style="text-align:right;"|יש<ref group=II>יש: Mo30 ויש< | + | |style="text-align:right;"|יש לד' שבטור השפל לקחת מן השלישי שבטור העליון למען כי שלישי הוא ולא נוכל כי השלישי שבטור העליון גלגל הוא נשיב מן הה' שהנחנו על הד' אחד על הגלגל יהיו י' יקח ד' ישארו ו' על הגלגל‫<ref group=II>יש: Mo30 ויש<br> |
| + | לד‫': Mo30 לך<br> | ||
| + | שבטור: Mo30 בטור<br> | ||
| + | למען כי שלישי הוא: Mo30; V398 om.<br> | ||
| + | השלישי: V397 <sup>ה</sup>שלישי <s>הוא</s><br> | ||
| + | גלגל הוא: Mo30 הוא גלגל<br> | ||
| + | נשיב: V397 נשוב<br> | ||
| + | על הד' אחד על הגלגל: Mo30 על הד' על הגלגל על הד' על הגלגל marg. אחד<br> | ||
| + | יקח: Mo30 נקח<br> | ||
| + | ישארו: Mo30 וישארו</ref> | ||
|- | |- | ||
| | | | ||
:*The 5 that is the beginning of the bottom row, which is fourth, takes from the fourth in the upper row, which is 9; 4 remain on the 9. | :*The 5 that is the beginning of the bottom row, which is fourth, takes from the fourth in the upper row, which is 9; 4 remain on the 9. | ||
| − | |style="text-align:right;"|יקח הה'<ref group=II>הה': V397 ה'< | + | |style="text-align:right;"|יקח הה' שהוא ראש בטור השפל והוא רביעי יקח מהרביעי שבטור העליון שהוא ט' ישארו על ט' ד‫'‫<ref group=II>הה‫': V397 ה‫'<br> |
| + | רביעי: V397 רביעית<br> | ||
| + | יקח: V397 om.<br> | ||
| + | שבטור: V397 שהוא בטור<br> | ||
| + | ישארו: Mo30; V397 marg. ישאר<br> | ||
| + | ט‫': Mo30 הט‫'</ref> | ||
|- | |- | ||
| | | | ||
:We divide again, because not everything has been taken out yet. The remainders from the top row are the 3, which is the first and fifth, 4 on the 9, 6 on the zero, 4 on the 4, and 2 on the 5. | :We divide again, because not everything has been taken out yet. The remainders from the top row are the 3, which is the first and fifth, 4 on the 9, 6 on the zero, 4 on the 4, and 2 on the 5. | ||
| − | |style="text-align:right;"|נשוב | + | |style="text-align:right;"|נשוב לחלק כי עדין לא יצא והנשארים ג' מן הטור העליון שהוא ראשון וחמישי ועל הט' ד' ועל הגלגל ו' ועל הד' ד' ועל הה' ב‫'‫<ref group=note>ב‫': Mo30 ב' וזו הב' הנשארת על הה' בטור העליון לא נקנה על הב' בטור השפל אי אפשר אלא נשיב אותה כלה אחורנית על הד' אצלה ויהיו כ"ד נחלקם על הב' בטור השפל לא תוכל לתת לו יותר מח' בשביל הבאים אחריו ואותה הח' תכתוב אותה במקומה ממעל לה' הראשנה שבטור השפל <s>וממעל</s> ומתחת הג' הראשנה שבטור העליון</ref>‫<ref group=II>נשוב: V397 שוב<br> |
| + | יצא: Mo30 יצא לחוץ<br> | ||
| + | מן הטור: Mo30; V397 מהטור<br> | ||
| + | וחמישי: Mo30 marg.<br> | ||
| + | ועל הט' ד‫': Mo30 וד' על הט‫'</ref> | ||
|- | |- | ||
| colspan=2| | | colspan=2| | ||
| Line 1,661: | Line 2,995: | ||
| | | | ||
:*We return the 2 back on the 4; they are 24. We give 8 to the 2, which is the fourth in the lower row; 8 remain on the 4. | :*We return the 2 back on the 4; they are 24. We give 8 to the 2, which is the fourth in the lower row; 8 remain on the 4. | ||
| − | |style="text-align:right;"|נשיב | + | |style="text-align:right;"|נשיב הב' על הד' והם כ"ד נתן לב' שהוא רביעי שבטור השפל ח' נשארו ח' על הד‫'‫<ref group=II>נשיב: V397 ונשים<br> |
| + | נתן: V397 וכן<br> | ||
| + | שבטור: Mo30 בטור<br> | ||
| + | ח‫': V397 ה‫'<br> | ||
| + | נשיב הב' ... ח‫': Mo30 marg.<br> | ||
| + | נשארו: Mo30 וישארו</ref> | ||
|- | |- | ||
| | | | ||
:*We return 7 of them back on the 6, and 1 remains on the 4; they are 76. We take 72 for the 9; 4 remain instead of the 6. | :*We return 7 of them back on the 6, and 1 remains on the 4; they are 76. We take 72 for the 9; 4 remain instead of the 6. | ||
| − | |style="text-align:right;"|נשיב<ref group=II>נשיב: V397 אם נאמר נשב< | + | |style="text-align:right;"|נשיב מהם ז' על הו' אחורנית וא' נשאר על הד' ויהיו ע"ו נקח לט' ע"ב נשארו ד' במקום הו‫'‫<ref group=II>נשיב: V397 אם נאמר נשב<br> |
| + | הו‫': Mo30; V398 ו‫'<br> | ||
| + | על הו' אחורנית: Mo30 marg. אחורנית על הו‫'<br> | ||
| + | ויהיו: V397 ויהיה<br> | ||
| + | נקח: V397 יקחו<br> | ||
| + | לט‫': V397 הט‫'<br> | ||
| + | נשארו: Mo30 ונשארו; V397 ישארו</ref> | ||
|- | |- | ||
| | | | ||
:*If we return 3 of them, the 4 in the lower row indeed can take from the 3 together with the 4 that follows it, which are 34, but only two will remain, and when we return it to the 3, they are only 23, whereas the 5 have to take 40. | :*If we return 3 of them, the 4 in the lower row indeed can take from the 3 together with the 4 that follows it, which are 34, but only two will remain, and when we return it to the 3, they are only 23, whereas the 5 have to take 40. | ||
| − | |style="text-align:right;"|אם<ref group=II>אם: Mo30 ואם< | + | |style="text-align:right;"|אם נשיב מהם ג' ואמת כי הד' שבטור השפל יוכלו לקחת מהג' עם הד' שאחריהם שהם ל"ד אך לא ישאר כי אם שנים וכשנשיב אותו על הג' יהיו כ"ג לבד ויש לה' שיקחו מ‫'‫<ref group=II>אם: Mo30 ואם<br> |
| + | ג‫': Mo30 om.<br> | ||
| + | ואמת: Mo30 באמת; V397 האמת<br> | ||
| + | שבטור: Mo30 בטור<br> | ||
| + | לקחת: V397 לקח<br> | ||
| + | מהג‫': Mo30 marg. מן הג‫'<br> | ||
| + | נשארו ח' על הד' ... שאחריהם: Mo30 twice - once on marg. and on the next page<br> | ||
| + | וכשנשיב: Mo30; V398 ואם נשיב<br> | ||
| + | הג‫': Mo30 ג‫'<br> | ||
| + | יהיו כ"ג לבד: V397 לא יהיו בם כ"ד<br> | ||
| + | לה‫': Mo30 לה' שבטור השפל<br> | ||
| + | שיקחו: Mo30 ליקח<br> | ||
| + | מ‫': Mo30 ד‫'</ref> | ||
|- | |- | ||
| | | | ||
::Therefore, we return the whole 4 back to the 4 and write a zero instead of the 4 corresponding to the zero in the upper row; they are 44. The 4 take 32; 12 remain. | ::Therefore, we return the whole 4 back to the 4 and write a zero instead of the 4 corresponding to the zero in the upper row; they are 44. The 4 take 32; 12 remain. | ||
| − | |style="text-align:right;"|לפיכך נשיב כל הד' אחורנית על הד' ונכתוב גלגל במקום הו' כנגד הגלגל<ref group=II>הגלגל: Mo30 הג'; V397 גלגל< | + | |style="text-align:right;"|לפיכך נשיב כל הד' אחורנית על הד' ונכתוב גלגל במקום הו' כנגד הגלגל שבטור העליון והם מ"ד יקחו הד' ל"ב נשארו י"ב‫<ref group=II>הגלגל: Mo30 הג'; V397 גלגל<br> |
| + | יקחו: Mo30 נקח<br> | ||
| + | הד' ל"ב: Mo30 לד' שבטור השפל<br> | ||
| + | נשארו: Mo30 וישארו; V397 ישארו</ref> | ||
|- | |- | ||
| | | | ||
:*We take 4 from them, because less is not enough for us; 8 remain on the 4 that are on the 9. With the 3 they are 43. The 5 take from 40. | :*We take 4 from them, because less is not enough for us; 8 remain on the 4 that are on the 9. With the 3 they are 43. The 5 take from 40. | ||
| − | |style="text-align:right;"|נקח מהם ד'<ref group=II>ד': Mo30 <sup>ד'</sup> ונשיבם אחורנית על הג' הראשנה שבטור הראשנה כדי לחלקו על ה' הראשנה שבטור השפל וישאר ח' במעלה השניה< | + | |style="text-align:right;"|נקח מהם ד' כי לא יהיה די לנו בפחות וישארו ח' על הד' שהם על הט' ועם הג' הם מ"ג יקחו הה' מ‫'‫<ref group=II>ד‫': Mo30 <sup>ד‫'</sup> ונשיבם אחורנית על הג' הראשנה שבטור הראשנה כדי לחלקו על ה' הראשנה שבטור השפל וישאר ח' במעלה השניה<br> |
| + | די: Mo30 om.<br> | ||
| + | די לנו: V398 לנו די<br> | ||
| + | ועם: Mo30 והד' עם<br> | ||
| + | כי לא יהיה ... הג‫': Mo30 marg.<br> | ||
| + | הם: Mo30 ויהיה<br> | ||
| + | מ"ג: Mo30 מ"ג בראשנה שבטור העליון<br> | ||
| + | יקחו: Mo30 תן<br> | ||
| + | הה' מ‫': Mo30 המ' על הה' שבטור השפל</ref> | ||
|- | |- | ||
| | | | ||
:3 remain on the 3, 8 on the 9, which are 80, a zero, which takes the number out of the hundreds and into the thousands, and 1 on the fourth that is on the 4, which is one thousand. These cannot be divided, because the divisor is greater than it; this is 2 thousand 9 hundred and 45. | :3 remain on the 3, 8 on the 9, which are 80, a zero, which takes the number out of the hundreds and into the thousands, and 1 on the fourth that is on the 4, which is one thousand. These cannot be divided, because the divisor is greater than it; this is 2 thousand 9 hundred and 45. | ||
| − | |style="text-align:right;"|ישארו<ref group=II>ישארו: Mo30 ישאר< | + | |style="text-align:right;"|ישארו ג' על הג' וח' על הט' שהם פ' וגלגל להוציאו ממאות ולהכניסו לאלפים וא' על הרביעי שהוא על הד' שהוא אלף ואלה לא יתחלקו כי המחולק גדול מזה שהוא אלפים וט' מאות ומ"ה‫<ref group=II>ישארו: Mo30 ישאר<br> |
| + | על: V398 ועל<br> | ||
| + | הט‫': V397 ט‫'<br> | ||
| + | לאלפים: V397 באלפים<br> | ||
| + | וא' על: V397 ואחר<br> | ||
| + | הד‫': V397 ד‫'<br> | ||
| + | ג' ...אלף: Mo30 ג' במעלה הראשנה בטור העליון וח' במעלה שניה וא' במעלה רביעית<br> | ||
| + | וט' מאות: V397 ותתקמ"ה</ref> | ||
|- | |- | ||
| | | | ||
*{{#annot:68921÷7053|157|eXVh}}Another example: we wish to divide 68 thousands, 9 hundred and 21 by 7 thousands and 53. | *{{#annot:68921÷7053|157|eXVh}}Another example: we wish to divide 68 thousands, 9 hundred and 21 by 7 thousands and 53. | ||
:<math>\scriptstyle68921\div7053</math> | :<math>\scriptstyle68921\div7053</math> | ||
| − | |style="text-align:right;"|<big>דמיון אחר</big> בקשנו לחלק ס"ח אלפים<ref group=II>ס"ח אלפים: V398 ששים אלף וח' אלפים< | + | |style="text-align:right;"|<big>דמיון אחר</big> בקשנו לחלק ס"ח אלפים וט' מאות וכ"א על ז' אלפים ונ"ג{{#annotend:eXVh}}‫<ref group=II>ס"ח אלפים: V398 ששים אלף וח' אלפים<br> |
| + | וט' מאות וכ"א: V397 ותתקכ"א<br> | ||
| + | ונ"ג: V397 נ"ג</ref> | ||
|- | |- | ||
| | | | ||
| Line 1,746: | Line 3,123: | ||
| | | | ||
:*The 7, which is fourth in the bottom row, cannot take from the 6, which is the fifth in the top row, since we cannot give the 7 what it needs. | :*The 7, which is fourth in the bottom row, cannot take from the 6, which is the fifth in the top row, since we cannot give the 7 what it needs. | ||
| − | |style="text-align:right;"|ז'<ref group=II>ז': Mo30 והנה ז'; V397 ... illegible< | + | |style="text-align:right;"|ז' שהוא רביעי בטור השפל לא יוכל לקחת מו' שהוא חמישי בטור העליון ומאחר שלא נוכל לתת לז' כל הצורך‫<ref group=II>ז‫': Mo30 והנה ז‫'; V397 ... illegible<br> |
| + | רביעי: V397 marg. הרביעי<br> | ||
| + | יוכל: Mo30; V397 נוכל<br> | ||
| + | מו‫': Mo30 מן הו‫'<br> | ||
| + | ומאחר: Mo30 ואח"כ אחר marg. וא"כ; V397 ... illegible; V398 אחר<br> | ||
| + | נוכל: Mo30 תוכל; V398 יוכל<br> | ||
| + | כל: V397; V398 om.<br> | ||
| + | הצורך: Mo30 צרכה</ref> | ||
|- | |- | ||
| | | | ||
:*We return all of it back on the 8; they are 68. So, from the 8, which is the fourth in the top row, we give 9 to the 7; we take it out on the 3, which is the fourth in the bottom row; so, 5 remain on the 8. | :*We return all of it back on the 8; they are 68. So, from the 8, which is the fourth in the top row, we give 9 to the 7; we take it out on the 3, which is the fourth in the bottom row; so, 5 remain on the 8. | ||
| − | |style="text-align:right;"|נשיב אותו<ref group=II>אותו: Mo30 הו'< | + | |style="text-align:right;"|נשיב אותו כלו אחורנית על הח' ויהיו ס"ח הנה חלקנו מן הח' שהוא רביעי לטור העליון נתן לז' ט' נוציאנו לחוץ על הג' שהוא רביעי בטור השפל נשארו ה' על הח‫'‫<ref group=II>אותו: Mo30 הו‫'<br> |
| + | כלו: Mo30 אלו<br> | ||
| + | כלו אחורנית: V397 אחורנית כלו<br> | ||
| + | ויהיו: V397 שהוא<br> | ||
| + | הנה: Mo30 והנה<br> | ||
| + | מן הח‫': V397 מהח‫'<br> | ||
| + | חלקנו ... העליון: Mo30 om.<br> | ||
| + | לז‫': Mo30 לה<br> | ||
| + | ט‫': Mo30 ט' והנה ז' פעמים ט' הם ס"ג וזו הט‫'<br> | ||
| + | נוציאנו: Mo30 נכתוב אותה; V398 נוציא לו<br> | ||
| + | לחוץ: Mo30 בחוץ<br> | ||
| + | הג‫': V398 ג‫'<br> | ||
| + | שהוא רביעי בטור: Mo30 שבטור<br> | ||
| + | נשארו: Mo30 נמצא שהיא עומדת במעלה ראשנה וישאר<br> | ||
| + | ה' על הח‫': Mo30 על הח' ה‫'</ref> | ||
|- | |- | ||
| | | | ||
:*The second to the 7 does not take anything because it is a zero. | :*The second to the 7 does not take anything because it is a zero. | ||
| − | |style="text-align:right;"|הנה<ref group=II>הנה: Mo30 והנה< | + | |style="text-align:right;"|הנה השני לז' לא יקח מאומה כי הוא גלגל‫<ref group=II>הנה: Mo30 והנה<br> |
| + | מאומה: Mo30 מה' מאומה</ref> | ||
|- | |- | ||
| | | | ||
:*The 5 must take from the 2, the fourth in the top row, which is the third in the division, but cannot take it. | :*The 5 must take from the 2, the fourth in the top row, which is the third in the division, but cannot take it. | ||
| − | |style="text-align:right;"|יש לה'<ref group=II>יש לה': Mo30 והנה הה' בטור השפל יש לנו< | + | |style="text-align:right;"|יש לה' שיקח מן ב' הרביעי בטור העליון שהוא שלישי לחלוק לא יוכל לקחת‫<ref group=II>יש לה‫': Mo30 והנה הה' בטור השפל יש לנו<br> |
| + | שיקח: Mo30 ליקח<br> | ||
| + | ב‫': V398 הב‫'<br> | ||
| + | מן ב' הרביעי: V397 מהרביעי ... illegible<br> | ||
| + | לחלוק: Mo30 בחלוק<br> | ||
| + | לא: Mo30; V397 ולא<br> | ||
| + | יוכל: Mo30; V398 נוכל</ref> | ||
|- | |- | ||
| | | | ||
:*So, we take 5 from the 9 that follows it. We return it back on the 2; they are 52, and 4 remain on the 9. The 5 take 45 out of 52; 7 remain on the 2. | :*So, we take 5 from the 9 that follows it. We return it back on the 2; they are 52, and 4 remain on the 9. The 5 take 45 out of 52; 7 remain on the 2. | ||
| − | |style="text-align:right;"|נקח<ref group=II>לקחת נקח: Mo30 אלא ליקח< | + | |style="text-align:right;"|נקח מן הט' שאחריו ה' נשיבם על הב' יהיו נ"ב וד' נשאר על הט' והה' מנ"ב יקחו מ"ה ישארו ז' על הב‫'‫<ref group=II>לקחת נקח: Mo30 אלא ליקח<br> |
| + | נשיבם: Mo30 ונשיבם אחור; V397 נשיבהו; V398 נשיב אותם<br> | ||
| + | הב‫': V398 ב‫'<br> | ||
| + | יהיו: Mo30 ויהיו<br> | ||
| + | נ"ב: V397 om.<br> | ||
| + | נשאר: V398 שנשאר<br>וד' נשאר: V397 נשאר ד‫'<br> | ||
| + | מנ"ב: V398 מהנ"ב<br> | ||
| + | והה' מנ"ב יקחו מ"ה: Mo30 ומאלה ג"כ ‫[marg. נ"ב]‫ תתן מ"ה לה' שבטור השפל<br> | ||
| + | ישארו: Mo30 וישארו<br> | ||
| + | על הב‫': V397 om.</ref> | ||
|- | |- | ||
| | | | ||
:*We take 3 from the 7 and return them back on the 1; they are 31. The 3 take 27; 4 remain on the 1. | :*We take 3 from the 7 and return them back on the 1; they are 31. The 3 take 27; 4 remain on the 1. | ||
| − | |style="text-align:right;"|מן<ref group=II>מן: Mo30 ומזו; V398 ומן< | + | |style="text-align:right;"|מן הז' נקח ג' ונשיבם אחורנית על א' והם ל"א יקחו ג' כ"ז נשאר ד' על א‫'‫<ref group=II>מן: Mo30 ומזו; V398 ומן<br> |
| + | ונשיבם: V398 נשיבם<br> | ||
| + | א‫': Mo30 הא' הראשנה שבטור העליון<br> | ||
| + | והם: Mo30 ויהיו<br> | ||
| + | כ"ז: V397 ט' marg. כ"ז<br> | ||
| + | יקחו ג' כ"ז: Mo30 מהם תתן כ"ז לג' שבטור השפל<br> | ||
| + | נשאר: Mo30 וישאר<br> | ||
| + | א‫': Mo30 הא' הראשנה שבטור העליון וד' במעלה שניה וד' <s>וא'</s> במעלה שלישית וה' במעלה רביעית בלי חלוק כי טור השפל יותר ממנו</ref> | ||
|- | |- | ||
| | | | ||
*{{#annot:680402÷2009|157|q1Q1}}Another example: we wish to divide 680402 by 2009. | *{{#annot:680402÷2009|157|q1Q1}}Another example: we wish to divide 680402 by 2009. | ||
:<math>\scriptstyle680402\div2009</math> | :<math>\scriptstyle680402\div2009</math> | ||
| − | |style="text-align:right;"|<big>דמיון אחר</big><ref group=II>אחר: V398 om.< | + | |style="text-align:right;"|<big>דמיון אחר</big> נרצה לחלק תר"פ אלפים ות"ב על אלפים וט‫'{{#annotend:q1Q1}}‫<ref group=II>אחר: V398 om.<br> |
| + | נרצה: V397 רצינו<br> | ||
| + | תר"פ אלפים ות"ב על אלפים וט‫': Mo30; V397; V398 om.</ref> | ||
|- | |- | ||
| | | | ||
| Line 1,816: | Line 3,239: | ||
| | | | ||
:Digits by digits so that there are 2 zeros in the top row and in the bottom row. | :Digits by digits so that there are 2 zeros in the top row and in the bottom row. | ||
| − | |style="text-align:right;"|מספרים על מספרים<ref group=II>על מספרים: Mo30 om.< | + | |style="text-align:right;"|מספרים על מספרים שיהיה בטור העליון ב' גלגלים וכן בטור השפל‫<ref group=II>על מספרים: Mo30 om.<br> |
| + | שיהיה: Mo30 שיהיו<br> | ||
| + | וד' במעלה שניה ... בטור העליון: Mo30 twice<br> | ||
| + | ב‫': Mo30 om.</ref> | ||
|- | |- | ||
| | | | ||
:*We give the 2 that is the fourth in the bottom row, 3 from the 6 that is the sixth in the top row. | :*We give the 2 that is the fourth in the bottom row, 3 from the 6 that is the sixth in the top row. | ||
| − | |style="text-align:right;"|נתן<ref group=II>נתן: Mo30 תן< | + | |style="text-align:right;"|נתן לב' הרביעי בטור השפל ג' מהו' הששי בטור העליון‫<ref group=II>נתן: Mo30 תן<br> |
| + | בטור: Mo30 שבטור<br> | ||
| + | הששי: V397 om.<br> | ||
| + | בטור: V397 שבטור</ref> | ||
|- | |- | ||
| | | | ||
::The zero that is the second in the bottom row has to take from the 8, but it takes nothing. | ::The zero that is the second in the bottom row has to take from the 8, but it takes nothing. | ||
| − | |style="text-align:right;"|הנה<ref group=II>הנה: Mo30 והנה< | + | |style="text-align:right;"|הנה הגלגל שני בטור השפל יש לו שיקח מן הח' ולא יקח כלום‫<ref group=II>הנה: Mo30 והנה<br> |
| + | שני: Mo30 השני<br> | ||
| + | שיקח: Mo30 ליקח</ref> | ||
|- | |- | ||
| | | | ||
::The zero that is the third in the bottom row has to take from the zero that is the third in the top row, but cannot take. | ::The zero that is the third in the bottom row has to take from the zero that is the third in the top row, but cannot take. | ||
| − | |style="text-align:right;"|והגלגל השלישי שבטור השפל יש לו שיקח<ref group=II>שיקח: Mo30 ליקח< | + | |style="text-align:right;"|והגלגל השלישי שבטור השפל יש לו שיקח מן הגלגל השלישי בטור העליון ולא יוכל לקחת‫<ref group=II>שיקח: Mo30 ליקח<br> |
| + | הגלגל: Mo30; V398 om.<br> | ||
| + | בטור: Mo30 שבטור<br> | ||
| + | יוכל: Mo30 <s>יון</s> יוכל</ref> | ||
|- | |- | ||
| | | | ||
:*The 9 that is the first in the bottom row must take from the 4 in the top row but cannot. Therefore, we have to return from the 8 that is the second in the top row, what is enough for it. We return 1 back, because it is enough for us. We write 7 on the 8, and the 1 that we have returned back on zero; it is taken as tens for us. That is still not enough. We take from them 3, return them back; 7 remain on the zero, and with the 3 they are 30 on the 4. | :*The 9 that is the first in the bottom row must take from the 4 in the top row but cannot. Therefore, we have to return from the 8 that is the second in the top row, what is enough for it. We return 1 back, because it is enough for us. We write 7 on the 8, and the 1 that we have returned back on zero; it is taken as tens for us. That is still not enough. We take from them 3, return them back; 7 remain on the zero, and with the 3 they are 30 on the 4. | ||
| − | |style="text-align:right;"|יש<ref group=II>יש: Mo30 ויש< | + | |style="text-align:right;"|יש לט' הראשון בטור השפל לקחת מד' שבטור העליון לא יוכל צריכין אנו שנשיב מן הח' השני בטור העליון מה שיספיק לו נשיב א' אחורנית כי די לנו בא' ונכתוב על הח' ז' והא' שהשיבונו אחורנית על הגלגל יצא לנו בעשרות ועוד לא יספיק נקח מהם ג' ונשיבם אחורנית ונשארו ז' על הגלגל והג' הם ל' על הד‫'‫<ref group=II>יש: Mo30 ויש<br> |
| + | הראשון: Mo30 הראשנה<br> | ||
| + | יש ... לקחת: V398 om.; marg. וט‫'<br> | ||
| + | לא: Mo30 ולא<br> | ||
| + | יוכל: V397 נוכל<br> | ||
| + | צריכין: V398 צריכים<br> | ||
| + | לו: Mo30 <s>ולא</s><br> | ||
| + | ונכתוב: V397 וכתוב<br> | ||
| + | שהשיבונו: V397 שהשבנו<br> | ||
| + | V398 marg. נ"ל שחסר מכאן<br> | ||
| + | והג‫': Mo30 וג‫'<br> | ||
| + | הם ל‫': Mo30 om.<br> | ||
| + | הד‫': Mo30 הד' והם ל"ד נקח <s>כ"א</s> marg. כ"ז; V398 הד' והם ל"ד נקח כ"ז</ref> | ||
|- | |- | ||
| | | | ||
:So, 7 remain instead of 4, as well as zero and two that are the first in the top row, 7 on the zero, and 7 on the 8. | :So, 7 remain instead of 4, as well as zero and two that are the first in the top row, 7 on the zero, and 7 on the 8. | ||
| − | |style="text-align:right;"|נשארו<ref group=II>נשארו: Mo30 שנשארו< | + | |style="text-align:right;"|נשארו ז' במקום ד' וגלגל ושנים הראשונים שבטור העליון וז' שעל הגלגל וז' שעל הח‫'‫<ref group=II>נשארו: Mo30 שנשארו<br> |
| + | ד‫': Mo30 הד‫'<br> | ||
| + | הראשונים: Mo30 ראשנים<br> | ||
| + | שבטור: Mo30 בטור<br> | ||
| + | שעל הגלגל וז‫': V397 om.</ref> | ||
|- | |- | ||
| | | | ||
:*We divide again, because not everything has been taken. We take 3 from the 7 that is on the 8 and put them beneath the first zero, which is fourth from the 8. | :*We divide again, because not everything has been taken. We take 3 from the 7 that is on the 8 and put them beneath the first zero, which is fourth from the 8. | ||
| − | |style="text-align:right;"|עוד נשוב<ref group=II>נשוב: V398 נשיב< | + | |style="text-align:right;"|עוד נשוב לחלק שהרי לא יצא נקח לו ג' מן הז' שעל הח' ונשימהו תחת הגלגל הראשון שהוא רביעי לח‫'‫<ref group=II>נשוב: V398 נשיב<br> |
| + | לא: Mo30 עדין לא<br> | ||
| + | יצא: Mo30 יצא לחוץ<br> | ||
| + | נקח: Mo30 נתן<br> | ||
| + | לו: Mo30 לב‫'<br> | ||
| + | מן ... ונשימהו: V397 om.</ref> | ||
|- | |- | ||
| | | | ||
::1 remains on the 8. | ::1 remains on the 8. | ||
| − | |style="text-align:right;"|ונשאר א' על הח‫'<ref group=II>הח': Mo30 ח'</ref> | + | |style="text-align:right;"|ונשאר א' על הח‫'<ref group=II>הח‫': Mo30 ח‫'</ref> |
|- | |- | ||
| | | | ||
:*The zero has to take the from the zero but cannot. | :*The zero has to take the from the zero but cannot. | ||
| − | |style="text-align:right;"|יש לגלגל שיקח מן הגלגל<ref group=II>הגלגל: V397 שעל הגלגל< | + | |style="text-align:right;"|יש לגלגל שיקח מן הגלגל לא יוכל‫<ref group=II>הגלגל: V397 שעל הגלגל<br> |
| + | יש לגלגל ... לא יוכל: Mo30 om.</ref> | ||
|- | |- | ||
| | | | ||
:*The zero that follows is has to take from its rank, which is the 7 on the 4 but cannot. | :*The zero that follows is has to take from its rank, which is the 7 on the 4 but cannot. | ||
| − | |style="text-align:right;"|ויש לגלגל<ref group=II>ויש לגלגל: Mo30 וגלגל< | + | |style="text-align:right;"|ויש לגלגל שאחריו שיקח ממעלתו שהיא הז' שעל הד' לא יוכל‫<ref group=II>ויש לגלגל: Mo30 וגלגל<br> |
| + | שאחריו: Mo30 om.; V397 שלאחריו<br> | ||
| + | שיקח: Mo30 יקח<br> | ||
| + | שהיא: Mo30; V397 שהוא<br> | ||
| + | שעל: Mo30; V398 על<br> | ||
| + | לא: Mo30 ולא</ref> | ||
|- | |- | ||
| | | | ||
:*The 9 must take from the zero that is in its rank, because it is the fourth in the division, but cannot, since there is nothing on the zero and it is impossible to return it back on the two, because the two is not in its rank. So, we return 3 from the 7 that is on the preceding 4 back to zero; they are 30. | :*The 9 must take from the zero that is in its rank, because it is the fourth in the division, but cannot, since there is nothing on the zero and it is impossible to return it back on the two, because the two is not in its rank. So, we return 3 from the 7 that is on the preceding 4 back to zero; they are 30. | ||
| − | |style="text-align:right;"|יש לט'<ref group=II>יש לט': Mo30 om.< | + | |style="text-align:right;"|יש לט' שיקח מן הגלגל שהוא מעלתו לפי שהוא רביעי לחלוק ולא יוכל לפי שאין על הגלגל כלום וגם לא יוכל להשיב אותו אחורנית על השנים כי השנים אינם מעלתו נשיב מן הז' שעל הד' שלפניו ג' נשימם על הגלגל והם ל‫'‫<ref group=II>יש לט‫': Mo30 om.<br> |
| + | שיקח: Mo30 ליקח<br> | ||
| + | מן הגלגל: V397 מהגלגל<br> | ||
| + | רביעי: Mo30 רביעית<br> | ||
| + | וגם לא: Mo30 ולא<br> | ||
| + | כי השנים אינם: Mo30 שאינם<br> | ||
| + | השנים כי השנים אינם: V397 illegible<br> | ||
| + | שעל: Mo30 על</ref> | ||
|- | |- | ||
| | | | ||
:3 remain on the zero, as well as 4 on the preceding 4, 7 on the zero that precedes the 8 and 1 on the 8. | :3 remain on the zero, as well as 4 on the preceding 4, 7 on the zero that precedes the 8 and 1 on the 8. | ||
| − | |style="text-align:right;"|נשארו ג' על הגלגל וד' על הד'<ref group=II>הד': V398 ד'< | + | |style="text-align:right;"|נשארו ג' על הגלגל וד' על הד' לפניו וז' על הגלגל שהוא לפני הח' וא' על הח‫'‫<ref group=II>הד‫': V398 ד‫'<br> |
| + | ג' נשימם ... הד' לפניו: Mo30 om.<br> | ||
| + | הח‫': Mo30 א‫'<br> | ||
| + | וא‫': Mo30 והא‫'</ref> | ||
|- | |- | ||
| | | | ||
:*We divide again because it is not all taken yet. The last in the lower row cannot take from the 1, so we return it back on the 7 that is on the zero; they are 17. We give it 8, take it out after the 3 that is the fourth in the division, because we divided from 7 that is on the zero. | :*We divide again because it is not all taken yet. The last in the lower row cannot take from the 1, so we return it back on the 7 that is on the zero; they are 17. We give it 8, take it out after the 3 that is the fourth in the division, because we divided from 7 that is on the zero. | ||
| − | |style="text-align:right;"|נשוב | + | |style="text-align:right;"|נשוב לחלק שעדין לא יצא לחוץ הנה מן הא' לא יוכל לקחת האחרון שבטור השפל נשיב אותו אחורנית על הז' שהוא על הגלגל והם י"ז נתן לו ח' נוציאם לחוץ אחרי הג' כי הוא רביעי לחלוק כי מן הז' שעל הגלגל חלקנו‫<ref group=II>נשוב: V398 נשיב<br> |
| + | שעדין: V397 שעוד<br> | ||
| + | הנה: Mo30 והנה<br> | ||
| + | לקחת: V397 ליקח<br> | ||
| + | והם: Mo30 שהוא<br> | ||
| + | ח‫': Mo30 ב' פעמים ח‫' marg. יהיו י"ו<br> | ||
| + | אחרי: Mo30; V398 אחר<br> | ||
| + | הוא: Mo30 om.</ref> | ||
|- | |- | ||
| | | | ||
::1 remains there. | ::1 remains there. | ||
| − | |style="text-align:right;"|ושם נשאר<ref group=II>נשאר: V397 נשאר לנו</ref> | + | |style="text-align:right;"|ושם נשאר א‫'‫<ref group=II>נשאר: V397 נשאר לנו</ref> |
|- | |- | ||
| | | | ||
:*The zero has to take from the 4, but cannot take. | :*The zero has to take from the 4, but cannot take. | ||
| − | |style="text-align:right;"|הנה<ref group=II>הנה: Mo30 והנה< | + | |style="text-align:right;"|הנה יש לגלגל שיקח מן הד' ולא יקח‫<ref group=II>הנה: Mo30 והנה<br> |
| + | הנה יש: V397 ויש<br> | ||
| + | שיקח: Mo30 ליקח</ref> | ||
|- | |- | ||
| | | | ||
:*Also the other zero has to take from the 3 that is on the zero that is in the top row but cannot take. | :*Also the other zero has to take from the 3 that is on the zero that is in the top row but cannot take. | ||
| − | |style="text-align:right;"|גם | + | |style="text-align:right;"|גם יש לגלגל האחר שיקח מן הג' שעל הגלגל שבטור העליון ולא יקח‫<ref group=II>גם: V397 וגם<br> |
| + | מן הג‫': V397 מהג‫'<br> | ||
| + | שבטור: Mo30; V398 בטור<br> | ||
| + | יקח: V397 יקח ט‫'</ref> | ||
|- | |- | ||
| | | | ||
:*The 9 has to take from the 2, but cannot, so we return back. If we say to the 3 that is on the zero that to give to the 2 that is first to the zero, it does not have enough, because it only has 3. So, we take one from the 4, which replaces the 4, it is on the zero, with the 3 they are 13. We take 7 from them and return them back on the 2; they are 72. All is taken out by the 9. | :*The 9 has to take from the 2, but cannot, so we return back. If we say to the 3 that is on the zero that to give to the 2 that is first to the zero, it does not have enough, because it only has 3. So, we take one from the 4, which replaces the 4, it is on the zero, with the 3 they are 13. We take 7 from them and return them back on the 2; they are 72. All is taken out by the 9. | ||
| − | |style="text-align:right;"|ויש לט' שיקח<ref group=II>שיקח: V398 שיקחו< | + | |style="text-align:right;"|ויש לט' שיקח מן הב' ולא יוכל נשיב אחורנית אם נאמר לג' שעל הגלגל שיתן לב' הראשון לגלגל אין לו מה שיספיק לו כי אין לו אלא ג' נקח מן הד' שבמקום הד' אחד ויהיה על הגלגל עם הג' י"ג נקח מהם ז' ונשיבם על הב' והם ע"ב יצאו הכל בט‫'‫<ref group=II>שיקח: V398 שיקחו<br> |
| + | מן הב‫': V397 מהב‫'<br> | ||
| + | שיתן: Mo30; V398 om.<br> | ||
| + | לגלגל: Mo30; V398 om.<br> | ||
| + | שיתן ... לגלגל: V397 om.<br> | ||
| + | לו: Mo30 marg.<br> | ||
| + | אלא: V398 אלה<br> | ||
| + | על הגלגל: V398 לגלגל<br> | ||
| + | כי אין לו ... ויהיה על הגלגל: Mo30 om.<br> | ||
| + | הכל: V397 על הכל</ref> | ||
|- | |- | ||
| | | | ||
:We write a zero on the 2, because there is nothing left on it; 6 are left on the zero that is second to the 2, 3 on the 4 and 1 on the zero that is second to the 8. These remain as they cannot be divided, because the divisor is greater from them. The remainder is one thousand 3 hundred and 60 and the divisor two thousand and 9. | :We write a zero on the 2, because there is nothing left on it; 6 are left on the zero that is second to the 2, 3 on the 4 and 1 on the zero that is second to the 8. These remain as they cannot be divided, because the divisor is greater from them. The remainder is one thousand 3 hundred and 60 and the divisor two thousand and 9. | ||
| − | |style="text-align:right;"|ונכתוב | + | |style="text-align:right;"|ונכתוב גלגל על הב' שלא נשאר עליו כלום ועל הגלגל שהוא שני לב' נשארו ו' וג' על ד' וא' על הגלגל שהוא שני לח' ואלה נשארו שלא יתחלקו כי המחולק גדול מזה כי המספר הנשאר אלף וג' מאות וס' והמחולק עליו הוא אלפים וט‫'‫<ref group=II>ונכתוב: Mo30 ותכתוב<br> |
| + | כלום: V397 כלל<br> | ||
| + | לב‫': Mo30 marg.<br> | ||
| + | נשארו ו' וג‫': Mo30 om.<br> | ||
| + | ד‫': Mo30 הז‫'; V397 הד‫'<br> | ||
| + | וא‫': Mo30 ואם<br> | ||
| + | שהוא: Mo30; V398 om.<br> | ||
| + | שני: Mo30 om.<br> | ||
| + | נשארו: Mo30 ישאר<br> | ||
| + | אלף: Mo30 הוא אלף; V398 הוא א‫' marg. אלף<br> | ||
| + | וג' מאות וס‫': V397 וש"ס</ref> | ||
|- | |- | ||
| | | | ||
*Another example, which is more valuable and difficult than all previous calculations without zero. | *Another example, which is more valuable and difficult than all previous calculations without zero. | ||
| − | |style="text-align:right;"|'''דמיון אחר''' נכבד וקשה מכל החשבונים שתחתיו<ref group=II>שתחתיו: Mo30 om.< | + | |style="text-align:right;"|'''דמיון אחר''' נכבד וקשה מכל החשבונים שתחתיו מאין גלגל‫<ref group=II>שתחתיו: Mo30 om.<br> |
| + | מאין: V397 מעין</ref> | ||
|- | |- | ||
|First, I shall explain that whenever a digit is separated from a digit, meaning that a zero is written in between, as for example, 203, we do not say: we return the 2 or, as much is needed according to the digit in the lower row, back to the 3, because the zero is in the middle, so we return the 2 or the one back to the zero and then divide according to the rule. | |First, I shall explain that whenever a digit is separated from a digit, meaning that a zero is written in between, as for example, 203, we do not say: we return the 2 or, as much is needed according to the digit in the lower row, back to the 3, because the zero is in the middle, so we return the 2 or the one back to the zero and then divide according to the rule. | ||
| − | |style="text-align:right;"|ובראשונה אפרש<ref group=II> | + | |style="text-align:right;"|ובראשונה אפרש כי לעולם כשיתרחק חשבון מחשבון והטעם שיכתב גלגל באמצע כדמיון זה ג0ב ‫<ref group=II>MS St.P374 excerpt 2 begin.</ref>לא נאמר נשיב הב' אל הג' או כמה שיצטרך לפי החשבון שבטור השפל לפי שגלגל באמצע אך נשיב הב' או האחד אל הגלגל ואז נחלק כמשפט‫<ref group=II>אפרש: V397 נפרש<br> |
| + | כשיתרחק: V397 שיתרחק<br> | ||
| + | שיכתב: Mo30 שיכתוב; V397 כי שיכתוב<br> | ||
| + | כדמיון זה: Mo30 כזה הדמיון<br> | ||
| + | ג0ב: Mo30 om.<br> | ||
| + | הב‫': V397 om; Mo30 ב‫'; V398 שנים<br> | ||
| + | הג‫': Mo30 ג‫'<br> | ||
| + | כמה: Mo30; V397 כמו<br> | ||
| + | שיצטרך: Mo30; V398 שנצטרך<br> | ||
| + | החשבון: V398 חשבון<br> | ||
| + | הב‫': V397 ב‫'<br> | ||
| + | האחד: V397 א‫'<br> | ||
| + | אל: V397 על<br> | ||
| + | ואז נחלק כמשפט: Mo30; V397; V398 om.</ref> | ||
|- | |- | ||
|I shall tell you one rule for all the numbers you divide, whether they are many or a few: You always have to divide the upper number by the lower one, until the end of the digits when it has come to its end. If there is a zero above, it can no longer be divided. | |I shall tell you one rule for all the numbers you divide, whether they are many or a few: You always have to divide the upper number by the lower one, until the end of the digits when it has come to its end. If there is a zero above, it can no longer be divided. | ||
| Line 1,903: | Line 3,419: | ||
*{{#annot:777777777÷9999|157|qk7v}}Example: we wish to divide 9 sevens by 4 nines. | *{{#annot:777777777÷9999|157|qk7v}}Example: we wish to divide 9 sevens by 4 nines. | ||
:<math>\scriptstyle777777777\div9999</math> | :<math>\scriptstyle777777777\div9999</math> | ||
| − | |style="text-align:right;"|<big>דמיון</big> בקשנו לחלק חשבון | + | |style="text-align:right;"|<big>דמיון</big> בקשנו לחלק חשבון ט' שביעיות על ד' תשיעיות{{#annotend:qk7v}}‫<ref group=II>MS St.P569 excerpt 1 begin.</ref>‫<ref group=II>חשבון: Mo30 marg.; V397 om.<br> |
| + | שביעיות: V397 marg.</ref> | ||
|- | |- | ||
| | | | ||
| Line 1,980: | Line 3,497: | ||
| | | | ||
:First, I shall teach you how to divide after you see that the 7 is less than the 9. | :First, I shall teach you how to divide after you see that the 7 is less than the 9. | ||
| − | |style="text-align:right;"|הנה ראש כל דבר אוֹרְךָ<ref group=II>אורך: Mo30 אלמדך< | + | |style="text-align:right;"|הנה ראש כל דבר אוֹרְךָ איכה תחלק אחר שתראה שהז' פחות מהט‫'‫<ref group=II>אורך: Mo30 אלמדך<br> |
| + | איכה: Mo30 איך<br> | ||
| + | תחלק: V397 תחלה<br> | ||
| + | מהט‫': V397 התשעה</ref> | ||
|- | |- | ||
!First version | !First version | ||
| Line 1,987: | Line 3,507: | ||
| | | | ||
:*You have to return it back. This is the diagram. First, when you start dividing, give 7 to the 9 and write it back in the rank of the 4, which you are dividing, that is second to the 9. | :*You have to return it back. This is the diagram. First, when you start dividing, give 7 to the 9 and write it back in the rank of the 4, which you are dividing, that is second to the 9. | ||
| − | |style="text-align:right;"|תצטרך להשיבו אחורנית וזהו הדמיון<ref group=II>הדמיון: V397 הדמיון שאראה לך< | + | |style="text-align:right;"|תצטרך להשיבו אחורנית וזהו הדמיון ותחלה כשתחל לחלק תתן לט' ז' ותכתבנה אחורנית במעלת הד' שתחלק ממנו שהוא שני לט‫'‫<ref group=II>הדמיון: V397 הדמיון שאראה לך<br> |
| + | כשתחל: V397 תחלה שתחלק<br> | ||
| + | לט‫': V397 om.<br> | ||
| + | ותכתבנה אחורנית: V397 om.<br> | ||
| + | במעלת: V397 במעלה<br> | ||
| + | שתחלק: V397 שתחלוק; V398 שהחלוק</ref> | ||
|- | |- | ||
| | | | ||
::The remainder is 14. Proceed so, until 7 remain in the first rank of the division, likewise 7 in the second [rank] of the division, 8 in the third of the division and 4 in the fourth of the division. | ::The remainder is 14. Proceed so, until 7 remain in the first rank of the division, likewise 7 in the second [rank] of the division, 8 in the third of the division and 4 in the fourth of the division. | ||
| − | |style="text-align:right;"|והנשאר י"ד וכן תעשה עד שישאר במעלת א' לחלוק ז' וכן בשני לחלוק ז'<ref group=II>וכן תעשה ...לחלוק ז': V397 om.< | + | |style="text-align:right;"|והנשאר י"ד וכן תעשה עד שישאר במעלת א' לחלוק ז' וכן בשני לחלוק ז' ובשלישי לחלוק ח' וברביעי לחלוק ד‫'‫<ref group=II>וכן תעשה ...לחלוק ז‫': V397 om.<br> |
| + | ובשלישי: V397 ומהנשאר בשלישי</ref> | ||
|- | |- | ||
| | | | ||
:*We divide again: we return the 7 that is in the second [rank] back to the third. We divide from there and write 7 beneath the sixth 7, which is fourth of the division; the remainder is 14. Proceed so, until 7 remain, after it we write 8, then 5 and then 4. | :*We divide again: we return the 7 that is in the second [rank] back to the third. We divide from there and write 7 beneath the sixth 7, which is fourth of the division; the remainder is 14. Proceed so, until 7 remain, after it we write 8, then 5 and then 4. | ||
| − | |style="text-align:right;"|נשוב לחלק נשיב<ref group=II>נשיב: V397 נשוב< | + | |style="text-align:right;"|נשוב לחלק נשיב הז' שהוא בשני אל השלישי אחורנית ונחלק ממנו ונכתוב ז' תחת ז' ששי שהוא ד' לחלוק והנשאר י"ד וכן תעשה עד שישאר ז' ואחריו נכתוב ח' ואחריו ה' ואחריו ד‫'‫<ref group=II>נשיב: V397 נשוב<br> |
| + | הז‫': V397 om.; V398 ז‫'<br> | ||
| + | אחורנית: V397 om.<br> | ||
| + | ונחלק: V398 ומה ^ נחלק marg. ואשר<br> | ||
| + | ממנו: V398 om.<br> | ||
| + | ונחלק ממנו: V397 ומהג' נחלק<br> | ||
| + | ששי: V398 om.<br> | ||
| + | וכן תעשה עד שישאר ז‫': V397 om.<br> | ||
| + | ואחריו ה‫': V397 om.</ref> | ||
|- | |- | ||
| | | | ||
:*We divide again: we return the 7 that on the 8 and put it in the fourth rank; the remainder is 15 and what you leave is: 8, then 5, then 5 and then 4. Write 7 beneath the third that is the fourth of the division. | :*We divide again: we return the 7 that on the 8 and put it in the fourth rank; the remainder is 15 and what you leave is: 8, then 5, then 5 and then 4. Write 7 beneath the third that is the fourth of the division. | ||
| − | |style="text-align:right;"|נשוב | + | |style="text-align:right;"|נשוב לחלק נשיב ז' על הח' ונשים אותה במערכת ד' והנותר ט"ו ומה שתשאיר ח' ואחריו ה' ואחריו ה' ואחריו ד' ותכתוב ז' תחת השלישי שהוא הרביעי לחלוק‫<ref group=II>נשוב: V398 נשיב<br> |
| + | ז‫': V398 om.<br> | ||
| + | הח‫': V398 הז‫'<br> | ||
| + | ומה: V398 נשיב ז' אחורנית מה<br> | ||
| + | שתשאיר: V398 שנשאיר<br> | ||
| + | הרביעי: V398 השביעי<br> | ||
| + | לחלוק: V398 om.<br> | ||
| + | נשוב לחלק ... הרביעי לחלוק: V397 om.</ref> | ||
|- | |- | ||
| | | | ||
:*We divide again: we return the 8 back to the 5 and put 8 beneath the eighth, which is 7 that is the fourth of the division. 5 remain on 5, then 5, then 5 and then 5. | :*We divide again: we return the 8 back to the 5 and put 8 beneath the eighth, which is 7 that is the fourth of the division. 5 remain on 5, then 5, then 5 and then 5. | ||
| − | |style="text-align:right;"|נשוב לחלק נשיב | + | |style="text-align:right;"|נשוב לחלק נשיב ח' על ה' ונתן ח' תחת השמיני שהוא ז' רביעי לחלוק ישאר ה' על ה' ואחריו ה' ואחריו ה' ואחריו ה‫'‫<ref group=II>נשיב: V397 נשוב<br> |
| + | ח‫': V397 ה‫'<br> | ||
| + | השמיני: V397 שמיני; V398 השמינית<br> | ||
| + | ז‫': V398 om.<br> | ||
| + | רביעי: V397 השביעי<br> | ||
| + | ה' על ה‫': V397 על ה' י"ב; V398 על ה' ה‫'<br> | ||
| + | ואחריו: V397 ומה שתשאר<br> | ||
| + | ה‫': V397 י"ד<br> | ||
| + | ה‫': V397 ד‫'</ref> | ||
|- | |- | ||
| | | | ||
:*We divide again: we return the 5 back to the 5 and put 5 beneath 7 that is the ninth, which is the fourth of the division; the remainder is 10 and what you leave is: 5, then 5, then 6 and then 2. | :*We divide again: we return the 5 back to the 5 and put 5 beneath 7 that is the ninth, which is the fourth of the division; the remainder is 10 and what you leave is: 5, then 5, then 6 and then 2. | ||
| − | |style="text-align:right;"|נשוב לחלק נתן ה' על ה' אחורנית | + | |style="text-align:right;"|נשוב לחלק נתן ה' על ה' אחורנית ונתן ה' תחת ז' התשיעי והוא רביעי לחלוק והנשאר י' ומה שתשאיר ה' ואחריו ה' ואחריו ו' ואחריו ב‫'‫ |
| + | <ref group=II>אחורנית: V397 om.<br> | ||
| + | שתשאיר: V397 שתשאר; V398 שנשאיר<br> | ||
| + | ו‫': V397 ה‫'<br> | ||
| + | ב‫': V397 ג‫'</ref> | ||
|- | |- | ||
| | | | ||
:It cannot be divided further, because everything has already been removed with the 5 and because the remainder is 5 thousand 5 hundred and 62 and the divisor is 9 thousand 9 hundred and 99. The received number is 77 thousand 7 hundred and 85. | :It cannot be divided further, because everything has already been removed with the 5 and because the remainder is 5 thousand 5 hundred and 62 and the divisor is 9 thousand 9 hundred and 99. The received number is 77 thousand 7 hundred and 85. | ||
| − | |style="text-align:right;"|ועוד לא יתחלק כי כבר יצא | + | |style="text-align:right;"|ועוד לא יתחלק כי כבר יצא לחוץ בה' וכי הנותר הוא ה' אלפים וה' מאות וס"ב והמחולק עליו ט' אלפים וט' מאות וצ"ט והמקובל ע"ז אלף וז' מאות ופ"ה‫<ref group=II>יצא: V397 יצאנו<br> |
| + | וה' מאות וס"ב: V397 ותקס"ב<br> | ||
| + | והמחולק ... וצ"ט: V397 om.<br> | ||
| + | והמקובל: V397 והמקובל הוא<br> | ||
| + | אלף: V397 אלפים<br> | ||
| + | וז' מאות ופ"ה: V397 ותשפ"ה</ref> | ||
|- | |- | ||
| | | | ||
:In total of the division of the number occurred: three 7, three 8, three 4, nine 5, one 6 and one 2. | :In total of the division of the number occurred: three 7, three 8, three 4, nine 5, one 6 and one 2. | ||
| − | |style="text-align:right;"|וכלל חלוק החשבון ג'<ref group=II>ג': V397 ד'< | + | |style="text-align:right;"|וכלל חלוק החשבון ג' זיינין וג' חיתין וג' דלתין וט' ההין וו' אחת וב' אחת‫<ref group=II>ג‫': V397 ד‫'<br> |
| + | וג‫': V397 וד‫'<br> | ||
| + | וג‫': V397 וד‫'<br> | ||
| + | דלתין: V397 דלתין וב' זתין כמספר דלתין<br> | ||
| + | וו' אחת וב' אחת: V397 om.</ref> | ||
|- | |- | ||
| | | | ||
:The rule is that in all the divisions there were 4 [digits in the dividends] except the last one, where it decreased to 3 [digits]. | :The rule is that in all the divisions there were 4 [digits in the dividends] except the last one, where it decreased to 3 [digits]. | ||
| − | |style="text-align:right;"|והכלל כי עם<ref group=II>עם: V397 על< | + | |style="text-align:right;"|והכלל כי עם כל החלוקים ד' חוץ מן האחרון שנתמעט עד ג‫'‫<ref group=II>עם: V397 על<br> |
| + | כל: V398 om.<br> | ||
| + | חוץ: V398 om.</ref> | ||
|- | |- | ||
!Second version | !Second version | ||
| Line 2,025: | Line 3,589: | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|לכן שימהו לאחור לז' ויהיו ע"ז וחלקם על ט' ולא תוכל ליתן | + | |style="text-align:right;"|לכן שימהו לאחור לז' ויהיו ע"ז וחלקם על ט' ולא תוכל ליתן לו יותר מז' לצורך שאר המספר וישארו י"ד תניח מהם ז' לחלוק השני וז' תשימהו לאחור על הז' השלישי ויהיו ע"ז וחלקם על ט' השני וישארו י"ד תניח מהם ז' לחלוק שני והז' תשימהו לאחור אל הרביעי יהיו ע"ז וחלקם על הט' השלישי וישארו י"ד תניח מהם ח' לחלוק שני והו' תשימהו לאחור אל הז' החמישי ויהיו ס"ז וחלקם על הט' הרביעי וישארו ד‫'‫<ref group=II>ליתן: Mo30 לתת<br> |
| + | ז‫': Mo30 <s>ט‫'</s> marg. ז‫'<br> | ||
| + | שני: Mo30 השני<br> | ||
| + | על: Mo30 אל</ref> | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|נמצא שנשארו | + | |style="text-align:right;"|נמצא שנשארו לחלוק השני ז' ז' ז' ז' ז' ח' ז' ז‫'‫<ref group=II>שנשארו: Mo30 שישארו<br> |
| + | ז' ז' ז' ז' ז' ח' ז' ז‫': Mo30 om.</ref> | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|'''החלוק השני''' תשלח ז' לאחור ויהיו ע"ז וחלקם על הט' הראשון וישארו י"ד תניח מהם ז' לחלוק שלישי והז' שלחהו לח' שלפניו ויהיו ע"ח וחלקם על הט' השני ותן | + | |style="text-align:right;"|'''החלוק השני''' תשלח ז' לאחור ויהיו ע"ז וחלקם על הט' הראשון וישארו י"ד תניח מהם ז' לחלוק שלישי והז' שלחהו לח' שלפניו ויהיו ע"ח וחלקם על הט' השני ותן לו ז' כמו כן וישארו ט"ו תניח מהם ח' לחלוק שלישי וז' תשלחהו לאחור אל הד' ויהיו ע"ד וחלקם על הט' השלישי וישארו י"א תניח מהם ה' לחלוק שלישי והו' שלחהו אל הז' ויהיו ס"ז וחלקם על הט' הרביעי וישארו ד‫'‫<ref group=II>ותן: Mo30 וישארו י"ד ויתן</ref> |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|נמצא שנשארו לחלוק שלישי ז' ז' ז' ד' ה' ח' ז‫'<ref group=II>ז' ז' ז' ד' ה' ח' ז': Mo30 om.</ref> | + | |style="text-align:right;"|נמצא שנשארו לחלוק שלישי ז' ז' ז' ד' ה' ח' ז‫'<ref group=II>ז' ז' ז' ד' ה' ח' ז‫': Mo30 om.</ref> |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|'''החלוק השלישי''' | + | |style="text-align:right;"|'''החלוק השלישי''' תשיב ז' לאחור ויהיו ע"ח חלקם על ט' הראשון ותוכל לתת לו ז' ולא יותר וישארו ט"ו תניח מהם ח' לחלוק הרביעי והז' שימהו לאחור אל הה' ויהיו ע"ה וחלקם על ט' השני ותן לו ז' כמו כן וישארו י"ב תניח מהם ה' לחלוק רביעי והז' שימהו לאחור אל ד' ויהיו ע"ד וחלקם על ט' שלישי וישארו י"א תניח מהם ה' לחלוק הרביעי והו' שימהו אחורנית אל הז' ויהיו ס"ז וחלקם על הט' הרביעי וישארו ד‫'‫<ref group=II>השלישי: Mo30 בשלישי<br> |
| + | תשיב: Mo30 תשים<br> | ||
| + | ולא: Mo30 לא<br> | ||
| + | והז‫': Mo30 וז‫'<br> | ||
| + | הה‫': Mo30 הב‫'<br> | ||
| + | שימהו: Mo30 שימם<br> | ||
| + | ד‫': Mo30 הד‫'<br> | ||
| + | וחלקם: Mo30 וחלק<br> | ||
| + | הרביעי: Mo30 רביעי</ref> | ||
|- | |- | ||
| | | | ||
| Line 2,043: | Line 3,619: | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|'''חלוק הרביעי''' | + | |style="text-align:right;"|'''חלוק הרביעי''' שים ח' אחורנית על הה' ויהיו פ"ה תוכל לחלקם על הט' הראשון וליתן לו ח' וישארו י"ג תניח מהם ה' לחלוק חמישי והח' שלחהו אחורנית אל הה' ויהיו פ"ה וחלקם על הט' השני וישארו י"ג תניח מהם ה' לחלוק חמישי והח' שלחהו אחורנית אל הד' ויהיו פ"ד וחלקם על הט' השלישי ח' כמו כן וישארו י"ב תניח מהם ה' לחלוק חמישי והז' שלחהו לאחור אל הז' ויהיו ע"ז וחלקם על הט' הרביעי וישארו ה‫'‫<ref group=II>הרביעי: Mo30 רביעי<br> |
| + | ח': Mo30 om.<br> | ||
| + | אחורנית: Mo30 אחרונים<br> | ||
| + | חמישי: Mo30 החמישי<br> | ||
| + | והח‫': Mo30 וח‫'<br> | ||
| + | וחלקם: Mo30 וחלקם ח"ח ג"כ<br> | ||
| + | הט‫': Mo30 הטור<br> | ||
| + | חמישי: Mo30 <s>שישי</s> marg. ה‫'<br> | ||
| + | והח‫': Mo30 וח‫'<br> | ||
| + | הז‫': Mo30 הע‫'</ref> | ||
|- | |- | ||
| | | | ||
| Line 2,049: | Line 3,634: | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|'''חלוק חמישי''' שים ה' אחורנית ויהיו נ"ה תן מהם ה' על הט' הראשון | + | |style="text-align:right;"|'''חלוק חמישי''' שים ה' אחורנית ויהיו נ"ה תן מהם ה' על הט' הראשון וישארו י' תניח מהם ה' בלתי מחולקים והה' תשלח אחורנית אל הה' השלישי ויהיו נ"ה ותן על הט' השני ה' כמו כן וישארו י' תניח מהם ה' בלתי מחולקים והה' שלחהו אל ה' הרביעי ויהיו נ"ה ותן על הט' השלישי ה' כמו כן וישארו י' תניח מהם ו' בלתי מחולקים והד' שלחהו אל הז' ויהיו מ"ז וחלקם על הט' הרביעי ה' כמו כן‫<ref group=II>ה' על הט' הראשון: Mo30 על הט' הראשון <sup>ה‫'</sup><br> |
| + | והה‫': Mo30 וה‫'<br> | ||
| + | השלישי: Mo30 השלישית<br> | ||
| + | והה‫': Mo30 וה‫'<br> | ||
| + | שלחהו: Mo30 שלחם<br> | ||
| + | ה‫': Mo30 הה‫'<br> | ||
| + | כן: Mo30 ה‫'<br> | ||
| + | שלחהו: Mo30 שלחם</ref> | ||
|- | |- | ||
| | | | ||
| Line 2,058: | Line 3,650: | ||
|- | |- | ||
|We complete by knowing the test for whether you divided correctly. | |We complete by knowing the test for whether you divided correctly. | ||
| − | |style="text-align:right;"|והנה נשלים | + | |style="text-align:right;"|והנה נשלים לדעת המאזנים אם חלקת נכונה‫<ref group=II>נשלים: Mo30 נשלם<br> |
| + | אם חלקת נכונה: Mo30; V397; V398 om.</ref> | ||
|- | |- | ||
| | | | ||
| Line 2,066: | Line 3,659: | ||
| | | | ||
:*Know the scale of the number by which you have divided [= the divisor], whether it consists of one or more digits. | :*Know the scale of the number by which you have divided [= the divisor], whether it consists of one or more digits. | ||
| − | |style="text-align:right;"|דע מאזני המספר<ref group=II>המספר: V397 מספר< | + | |style="text-align:right;"|דע מאזני המספר שחלקת עליו בין שיהיה אחד או רבים‫<ref group=II>המספר: V397 מספר<br> |
| + | שיהיה: Mo30 שהיה</ref> | ||
|- | |- | ||
| | | | ||
:*Know also the scale of the quotient that you wrote between the two rows, whether it consists of one or more digits. | :*Know also the scale of the quotient that you wrote between the two rows, whether it consists of one or more digits. | ||
| − | |style="text-align:right;"|גם<ref group=II>גם: Mo30 ג"כ< | + | |style="text-align:right;"|גם דע מאזני המספר שעלה בחלוק שכתבת בין שני הטורים בין שיהיה אחד או רבים‫<ref group=II>גם: Mo30 ג"כ<br> |
| + | המספר: V397; V398 מספר<br> | ||
| + | שכתבת: Mo30; V397 שהוא</ref> | ||
|- | |- | ||
| | | | ||
:Multiply them by each other, the middle one by the lower one and know how much remains [after subtracting] the nines; this is the reserved, if you have no remainder that remains, because it is smaller than the divisor. | :Multiply them by each other, the middle one by the lower one and know how much remains [after subtracting] the nines; this is the reserved, if you have no remainder that remains, because it is smaller than the divisor. | ||
| − | |style="text-align:right;"|וכפול זה<ref group=II>זה: V397 marg.< | + | |style="text-align:right;"|וכפול זה על זה האמצעי על התחתון ודע כמה נשאר על ט' ט' והוא השמור אם לא נשאר לך מספר שנשאר שהוא פחות מהמספר שחלקת עליו‫<ref group=II>זה: V397 marg.<br> |
| + | האמצעי: V397 אמצעי; V398 ר"ל אמצעי<br> | ||
| + | נשאר: Mo30; V398 הנשאר<br> | ||
| + | ט'ט‫': Mo30 התשיעיות; V397 התשיעית; V398 תשיעית<br> | ||
| + | אם: Mo30 ואם<br> | ||
| + | שנשאר: V397 ששוי לחלק; Mo30; V398 שנשאר לחלק<br> | ||
| + | מהמספר: Mo30 המספר<br> | ||
| + | עליו: Mo30 עליו marg. לא תקח מאומה</ref> | ||
|- | |- | ||
| | | | ||
:For, if there is a remainder, take its scale and add it to the reserved that you have; the sum is the actual reserved. | :For, if there is a remainder, take its scale and add it to the reserved that you have; the sum is the actual reserved. | ||
| − | |style="text-align:right;"|כי<ref group=II>כי: Mo30 אבל< | + | |style="text-align:right;"|כי אם נשאר קח המאזנים שלו וחבר אותו עם השמור שהיה לך והמחובר הוא השמור באמת‫<ref group=II>כי: Mo30 אבל<br> |
| + | כי אם: V398 ואם<br> | ||
| + | קח: Mo30 מן<br> | ||
| + | וחבר: Mo30 תחבר</ref> | ||
|- | |- | ||
| | | | ||
:Look at the scale of the number that you have divided [= the dividend] that was in the top row, if it is equal to the scale of the reserved, you know that your calculation is correct. | :Look at the scale of the number that you have divided [= the dividend] that was in the top row, if it is equal to the scale of the reserved, you know that your calculation is correct. | ||
| − | |style="text-align:right;"|וראה מאזני המספר הגדול שחלקת אותו שהיה | + | |style="text-align:right;"|וראה מאזני המספר הגדול שחלקת אותו שהיה בטור העליון אם היה<ref group=II>MS St.P374 excerpt 2 end</ref> שוה למאזני השמור תדע כי חשבונך אמת‫<ref group=II>שהיה: V397 שיהיה<br> |
| + | אם: Mo30 ואם<br> | ||
| + | שוה למאזני: Mo30 כמאזני</ref> | ||
|- | |- | ||
| | | | ||
| Line 2,090: | Line 3,698: | ||
| | | | ||
:If you multiply the quotient by the divisor, then add to them the remainder from the division, then the sum is equal to the digits of the top row and the division is correct. | :If you multiply the quotient by the divisor, then add to them the remainder from the division, then the sum is equal to the digits of the top row and the division is correct. | ||
| − | |style="text-align:right;"|ואם תכפול מה<ref group=II>מה: V398 כל מה< | + | |style="text-align:right;"|ואם תכפול מה שיעלה בחלוק על המספר שחלקת עליו אחר שתחבר אליהם מה שנשאר לחלק אז יהיה המחובר שוה למספרי הטור העליון וחלוק נכון‫<ref group=II>מה: V398 כל מה<br> |
| + | שיעלה: V397 שתעלה; Mo30; V398 שעלה<br> | ||
| + | על: V398 על כל<br> | ||
| + | לחלק: V398 לחלוק<br> | ||
| + | למספרי: Mo30; V397 למספר<br> | ||
| + | וחלוק נכון: Mo30; V397; V398 om.</ref> | ||
|- | |- | ||
| − | !Additional excerpts<ref group=II>Mo30; V397 om.</ref> | + | !Additional excerpts‫<ref group=II>Mo30; V397 om.</ref> |
| | | | ||
|- | |- | ||
| Line 2,099: | Line 3,712: | ||
|- | |- | ||
|If the number you by which you divide [= the divisor] is two digits or more, divide the last of the top row by the last of the bottom row, if the digit of the top row is greater than that of the bottom [row]. | |If the number you by which you divide [= the divisor] is two digits or more, divide the last of the top row by the last of the bottom row, if the digit of the top row is greater than that of the bottom [row]. | ||
| − | |style="text-align:right;"|אם המספר שתחלק עליו שני מספרים או יותר חלק סוף הטור העליון<ref group=II>העליון: V398 om.</ref> | + | |style="text-align:right;"|אם המספר שתחלק עליו שני מספרים או יותר חלק סוף הטור העליון על סוף הטור השפל אם מספר העליון גדול מהשפל‫<ref group=II>העליון: V398 om.</ref> |
|- | |- | ||
|But if the lower one is greater than the upper one, return the last of the upper one back as tens to the preceding rank and add it to what is written there. | |But if the lower one is greater than the upper one, return the last of the upper one back as tens to the preceding rank and add it to what is written there. | ||
| Line 2,108: | Line 3,721: | ||
|- | |- | ||
|Take from there what you can give the last lower digit. Write down what you can give to all the other bottom digits, as what you give to the last, and write the quotient in the middle between the two rows, far from the rank you divided as the distance from the last bottom digit from the starting digit. | |Take from there what you can give the last lower digit. Write down what you can give to all the other bottom digits, as what you give to the last, and write the quotient in the middle between the two rows, far from the rank you divided as the distance from the last bottom digit from the starting digit. | ||
| − | |style="text-align:right;"|וקח ממנו מה שתוכל לתת למספר השפל האחרון וכתוב מה | + | |style="text-align:right;"|וקח ממנו מה שתוכל לתת למספר השפל האחרון וכתוב מה שתוכל לתת לכל מספרי השפל האחרים מה שתתן לאחרון והיוצא כתבהו באמצע שני הטורים רחוק מן המעלה שחלקת ממנה כמרחק סוף השפל מראשו‫<ref group=II>וכתוב מה: V398 ובתנאי</ref> |
|- | |- | ||
|Proceed like this for all the divisions, doing the same for them as for this division: count from the rank which you divided as the distance of the last bottom digit from its starting digit, and write the quotient there. Then, from the digit that is second to the last bottom digit take the digit that is next to the rank from which you divided, as much times as the number that is written between the rows. | |Proceed like this for all the divisions, doing the same for them as for this division: count from the rank which you divided as the distance of the last bottom digit from its starting digit, and write the quotient there. Then, from the digit that is second to the last bottom digit take the digit that is next to the rank from which you divided, as much times as the number that is written between the rows. | ||
| − | |style="text-align:right;"|וכן תעשה לכל החלוקים שתשוב עליהם בזה החלוק שתמנה מן המעלה שחלקת ממנה כמספר סוף השפל מראשו ושם תכתוב היוצא בחלוק ומהמספר<ref group=II>ומהמספר: V398 והמספר</ref> | + | |style="text-align:right;"|וכן תעשה לכל החלוקים שתשוב עליהם בזה החלוק שתמנה מן המעלה שחלקת ממנה כמספר סוף השפל מראשו ושם תכתוב היוצא בחלוק ומהמספר השני לסוף השפל תקחנו מן הסמוך למעלה שחלקת ממנו כמספר הכתוב באמצע הטורים‫<ref group=II>ומהמספר: V398 והמספר</ref> |
|- | |- | ||
|If it is not enough for you, return what is left of the rank that you first divided back to the preceding rank and add it to what is written in it, then take your number from it. Do this with all. | |If it is not enough for you, return what is left of the rank that you first divided back to the preceding rank and add it to what is written in it, then take your number from it. Do this with all. | ||
| − | |style="text-align:right;"|ואם לא יספיק לך תשיב | + | |style="text-align:right;"|ואם לא יספיק לך תשיב מה שנשאר מן המעלה שחלקת תחלה אחורנית אל המעלה הקודמת לה ותצרף עם מה שנכתב בה וקח ממנה מספרך וכן תעשה לכלם‫<ref group=II>תשיב: V398 השיב<br> |
| + | ותצרף: V398 ותצטרף</ref> | ||
|- | |- | ||
| | | | ||
| Line 2,123: | Line 3,737: | ||
| | | | ||
:*The 9 in the bottom row is greater than 8 that is the end of the top [row], so you return the 8 back to the 3; they are 83. We give 9 of the 83 to the 9; they are 81 and 2 are left on the 3. | :*The 9 in the bottom row is greater than 8 that is the end of the top [row], so you return the 8 back to the 3; they are 83. We give 9 of the 83 to the 9; they are 81 and 2 are left on the 3. | ||
| − | |style="text-align:right;"|הנה הט' שבטור השפל יותר | + | |style="text-align:right;"|הנה הט' שבטור השפל יותר מח' שהוא סוף העליון על כן תשיב הח' אחורנית על הג' יהיו פ"ג ונתן לט' מהפ"ג ט' יהיו פ"א ונשארו ב' על הג‫'‫<ref group=II>יותר: V398 יתר<br> |
| + | תשיב: V398 השיב</ref> | ||
|- | |- | ||
| | | | ||
| Line 2,131: | Line 3,746: | ||
| | | | ||
:*The bottom 3 cannot take from the top 5, because it is not of the same rank, so return three from 5 back to 2; they are 32. We subtract 27 from them, which are 9 times 3, which is the first of the lower [row]; 5 remain on the 2 and 2 on the 5. | :*The bottom 3 cannot take from the top 5, because it is not of the same rank, so return three from 5 back to 2; they are 32. We subtract 27 from them, which are 9 times 3, which is the first of the lower [row]; 5 remain on the 2 and 2 on the 5. | ||
| − | |style="text-align:right;"|והג' התחתון לא יוכל לקחת מן הה' העליון | + | |style="text-align:right;"|והג' התחתון לא יוכל לקחת מן הה' העליון כי אינו מעלתו לכן השיב מן הה' שלשה על הב' יהיו ל"ב נסיר מהם כ"ז שהם ט' פעמים ג' שהוא ראשון השפל ונשארו ה' על הב' וב' על הה‫'‫<ref group=II>והג' ... העליון: V398 om.<br> |
| + | כי: V398 כי marg. אע"פ שהוא</ref> | ||
|- | |- | ||
| | | | ||
::Since the lower 9 is in the third rank and 9 has already resulted in the division, we write it in the second rank, which is the third to the top 3 which you divided. | ::Since the lower 9 is in the third rank and 9 has already resulted in the division, we write it in the second rank, which is the third to the top 3 which you divided. | ||
| − | |style="text-align:right;"|ובעבור שהט' התחתון הוא במעלה השלישית וכבר יצא בחלוק ט' נכתבנו<ref group=II>נכתבנו: V398 תכתבהו</ref> | + | |style="text-align:right;"|ובעבור שהט' התחתון הוא במעלה השלישית וכבר יצא בחלוק ט' נכתבנו במעלה השנית שהוא שלישי לג' העליון שחלקת ממנו‫<ref group=II>נכתבנו: V398 תכתבהו</ref> |
|- | |- | ||
| | | | ||
:*We divide again and return the 2 that is on the 3 back to the 2 that is on the 5; they are 22. We subtract 9 twice from them, because 9 is the lower [digit]; 4 remain on the 5. We write the 2 in the first rank, which is the third to the top 5, which you divided in this division. | :*We divide again and return the 2 that is on the 3 back to the 2 that is on the 5; they are 22. We subtract 9 twice from them, because 9 is the lower [digit]; 4 remain on the 5. We write the 2 in the first rank, which is the third to the top 5, which you divided in this division. | ||
| − | |style="text-align:right;"|נשוב לחלק ונשיב ב' אשר על הג' אל הב' אשר על | + | |style="text-align:right;"|נשוב לחלק ונשיב ב' אשר על הג' אל הב' אשר על הה' יהיו כ"ב נסיר מהם ב' פעמים ט' כי ט' הוא השפל ונשארו ד' על הה' ונכתוב ב' במעלה הראשונה שהיא שלישית לה' העליון שחלקת ממנו בזה החלוק‫<ref group=II>על: V398 אל<br> |
| + | כי ט‫': V398 <s>כי ט‫'</s> כי ט‫'</ref> | ||
|- | |- | ||
| | | | ||
:*The zero cannot take anything from the 5 that is on the 2. | :*The zero cannot take anything from the 5 that is on the 2. | ||
| − | |style="text-align:right;"|הנה הגלגל לא יוכל לקחת מאומה מן הה' אשר על<ref group=II>אשר על: V398 שעל</ref> | + | |style="text-align:right;"|הנה הגלגל לא יוכל לקחת מאומה מן הה' אשר על הב‫'‫<ref group=II>אשר על: V398 שעל</ref> |
|- | |- | ||
| | | | ||
| Line 2,156: | Line 3,773: | ||
*{{#annot:11350÷110|157|SdNR}}Another example: we wish to divide 11350 by 110. | *{{#annot:11350÷110|157|SdNR}}Another example: we wish to divide 11350 by 110. | ||
:<math>\scriptstyle11350\div110</math> | :<math>\scriptstyle11350\div110</math> | ||
| − | |style="text-align:right;"|<big>דמיון אחר</big> רצינו לחלק י"א אלף וש"נ<ref group=II>וש"נ: V398 <s>ש"ה</s> marg. ש"נ</ref> | + | |style="text-align:right;"|<big>דמיון אחר</big> רצינו לחלק י"א אלף וש"נ על ק"י{{#annotend:SdNR}}‫<ref group=II>וש"נ: V398 <s>ש"ה</s> marg. ש"נ</ref> |
|- | |- | ||
| | | | ||
:*We take 1 from the last top 1 and write it in the third rank beneath the top 3; because the last lower 1 is in the third rank, so we remove the result from the last digit from which you started dividing. | :*We take 1 from the last top 1 and write it in the third rank beneath the top 3; because the last lower 1 is in the third rank, so we remove the result from the last digit from which you started dividing. | ||
| − | |style="text-align:right;"|לקחנו א'<ref group=II>א': V398 א' האחרון בטור השפל< | + | |style="text-align:right;"|לקחנו א' מן הא' האחרון העליון וכתבנוהו במעלה השלישית תחת הג' העליון לפי שא' האחרון השפל הוא במעלה השלישית וככה נרחיק היוצא מסוף המספר שהחלות לחלק ממנו‫<ref group=II>א‫': V398 א' האחרון בטור השפל<br> |
| + | העליון: V398 <s>בטור</s> העליון<br> | ||
| + | וכתבנוהו: V398 והנה א' וכתבנוהו<br> | ||
| + | המספר: V398 העליון</ref> | ||
|- | |- | ||
| | | | ||
| Line 2,184: | Line 3,804: | ||
| | | | ||
:For the second 1 in the bottom row, we take from the top 5; 2 are left on the 5 and these are twenty that cannot be divided. | :For the second 1 in the bottom row, we take from the top 5; 2 are left on the 5 and these are twenty that cannot be divided. | ||
| − | |style="text-align:right;"|וא' השני בטור השפל נקח מה' העליון ישארו ב' על הה'<ref group=II>הה': V398 ה | + | |style="text-align:right;"|וא' השני בטור השפל נקח מה' העליון ישארו ב' על הה' והם עשרים שלא יתחלקו‫<ref group=II>הה‫': V398 ה‫'<br> |
| + | יתחלקו: V398 נתחלקו</ref> | ||
|- | |- | ||
| | | | ||
| Line 2,205: | Line 3,826: | ||
|It is written in the books of the arithmeticians that he who wants to know how much is the sum of the numbers that proceed successively up to a known number, multiplies it by its half plus one half and the result is the sum. | |It is written in the books of the arithmeticians that he who wants to know how much is the sum of the numbers that proceed successively up to a known number, multiplies it by its half plus one half and the result is the sum. | ||
:<math>\scriptstyle\sum_{i=1}^{n} i=n\sdot\left[\left(\frac{1}{2}\sdot n\right)+\frac{1}{2}\right]</math> | :<math>\scriptstyle\sum_{i=1}^{n} i=n\sdot\left[\left(\frac{1}{2}\sdot n\right)+\frac{1}{2}\right]</math> | ||
| − | |style="text-align:right;"|כתוב בספרי<ref group=III>בספרי: V397 בשערי< | + | |style="text-align:right;"|כתוב בספרי חכמי החשבון כי הרוצה לדעת כמה המחובר מן המספרים שיעברו על הסדר עד סוף מספר ידוע יכפול אותו על חציו בתוספת חצי אחד והעולה הוא המחובר‫<ref group=III>בספרי: V397 בשערי<br> |
| + | כי: Mo30; V397 om.<br> | ||
| + | מן המספרים: Mo30 מהמספרי‫'<br> | ||
| + | שיעברו: Mo30; V397 שעברו<br> | ||
| + | יכפול: Mo30 כפול<br> | ||
| + | על חציו: Mo30 בחציו<br> | ||
| + | בתוספת: Mo30 גם בתוספת</ref> | ||
|- | |- | ||
| | | | ||
*{{#annot:1-11|669|OprP}}Example: we wish to know how much are the numbers that are summed from 1 to 11? | *{{#annot:1-11|669|OprP}}Example: we wish to know how much are the numbers that are summed from 1 to 11? | ||
:<math>\scriptstyle\sum_{i=1}^{11} i</math> | :<math>\scriptstyle\sum_{i=1}^{11} i</math> | ||
| − | |style="text-align:right;"|<big>דמיון</big> רצינו לדעת כמה הם<ref group=III>כמה הם: Mo30 om.< | + | |style="text-align:right;"|<big>דמיון</big> רצינו לדעת כמה הם המספרים המחוברים מא' עד סוף י"א{{#annotend:OprP}}‫<ref group=III>כמה הם: Mo30 om.<br> |
| + | המספרים: V397 מספרים<br> | ||
| + | המחוברים: Mo30 שהם; V397 marg.<br> | ||
| + | סוף: Mo30 om.</ref> | ||
|- | |- | ||
| | | | ||
:We know that the half of 11 is 5 and a half, we add to it one half, it is 6, then we multiply 11 by 6, they are 66 and this is the sum. | :We know that the half of 11 is 5 and a half, we add to it one half, it is 6, then we multiply 11 by 6, they are 66 and this is the sum. | ||
| − | |style="text-align:right;"|הנה ידענו | + | |style="text-align:right;"|הנה ידענו כי חצי י"א ה' וחצי נוסיף חצי אחד הנה יהיו ו' והנה נכפול י"א על ו' יעלו ס"ו והוא המחובר‫<ref group=III>ידענו: V397 ידע<br> |
| + | הנה: V397 om.<br> | ||
| + | הנה יהיו: Mo30 ויהיו<br> | ||
| + | והנה: Mo30 om.<br> | ||
| + | יעלו: Mo30; V397 עלו<br> | ||
| + | המחובר: Mo30 המבוקש</ref> | ||
|- | |- | ||
| colspan=2| | | colspan=2| | ||
| Line 2,222: | Line 3,857: | ||
*{{#annot:1-18|669|IeC7}}Another example for an even number [of terms]: how much is the sum up to 18? | *{{#annot:1-18|669|IeC7}}Another example for an even number [of terms]: how much is the sum up to 18? | ||
:<math>\scriptstyle\sum_{i=1}^{18} i</math> | :<math>\scriptstyle\sum_{i=1}^{18} i</math> | ||
| − | |style="text-align:right;"|<big>דמיון אחר</big> בזוגות כמה<ref group=III>כמה: Mo30 רצינו לדעת כמה< | + | |style="text-align:right;"|<big>דמיון אחר</big> בזוגות כמה המחובר עד סוף י"ח{{#annotend:IeC7}}‫<ref group=III>כמה: Mo30 רצינו לדעת כמה<br> |
| + | סוף: Mo30; V397 om.</ref> | ||
|- | |- | ||
| | | | ||
:We take its half, which is 9, multiply 18 by it and the result is 162, then we have to multiply 18 again by one half, the result is 9, add it to 162, the result is 171 and it is the sum. | :We take its half, which is 9, multiply 18 by it and the result is 162, then we have to multiply 18 again by one half, the result is 9, add it to 162, the result is 171 and it is the sum. | ||
| − | |style="text-align:right;"|והנה<ref group=III>והנה: V397 הנה< | + | |style="text-align:right;"|והנה לקחנו חציו והוא ט' כפלנו י"ח עליו ועלו קס"ב ועוד יש לכפול חשבון י"ח על חצי אחד יעלו ט' חבר אותו עם קס"ב יעלו קע"א והוא המחובר‫<ref group=III>והנה: V397 הנה<br> |
| + | והוא: V397 הם<br> | ||
| + | כפלנו: V397 כפלו<br> | ||
| + | ועלו: Mo30 עלו<br> | ||
| + | ועוד: V397 עוד<br> | ||
| + | יש: Mo30 יש לו; V397 יש לנו<br> | ||
| + | חשבון: Mo30; V397 om.<br> | ||
| + | יעלו: Mo30 יעלה<br> | ||
| + | חבר אותו: Mo30 חברם<br> | ||
| + | עם: Mo30 אל</ref> | ||
|- | |- | ||
| colspan=2| | | colspan=2| | ||
| Line 2,232: | Line 3,877: | ||
|- | |- | ||
|Every number is in accordance with these two ways. | |Every number is in accordance with these two ways. | ||
| − | |style="text-align:right;"|ועל אלו שני<ref group=III>אלו שני: Mo30 שני אלו< | + | |style="text-align:right;"|ועל אלו שני הדרכים הולך כל חשבון‫<ref group=III>אלו שני: Mo30 שני אלו<br> |
| + | הדרכים: V397 דרכים<br> | ||
| + | הולך: Mo30; V397 om.<br> | ||
| + | חשבון: Mo30 החשבון</ref> | ||
|- | |- | ||
|Another way: add one to the last number, multiply by half the number and the result is the sum. | |Another way: add one to the last number, multiply by half the number and the result is the sum. | ||
| Line 2,259: | Line 3,907: | ||
|- | |- | ||
|Abraham the author said: | |Abraham the author said: | ||
| − | |style="text-align:right;"|<big>אמר אברהם<ref group=III>אברהם: Mo30 אברהם אבן עזרא; V397 אברהם ן' עזרא</ref | + | |style="text-align:right;"|<big><div id="Abraham said">אמר אברהם המחבר</div></big>‫<ref group=III>אברהם: Mo30 אברהם אבן עזרא; V397 אברהם ן' עזרא</ref> |
|- | |- | ||
|I have found another way: add to the square of the last number the root that is the last number itself, then see how much is the sum and half the sum is the required. | |I have found another way: add to the square of the last number the root that is the last number itself, then see how much is the sum and half the sum is the required. | ||
:<math>\scriptstyle\sum_{i=1}^{n} i=\frac{1}{2}\sdot\left(n^2+n\right)</math> | :<math>\scriptstyle\sum_{i=1}^{n} i=\frac{1}{2}\sdot\left(n^2+n\right)</math> | ||
| − | |style="text-align:right;"|מצאתי דרך אחרת תוסיף<ref group=III>תוסיף: Mo30 שתוסיף; V397 שאוסיף< | + | |style="text-align:right;"|מצאתי דרך אחרת תוסיף על מרובע סוף החשבון השרש שהוא בעצמו וראה כמה המחובר וחצי המחובר הוא המבוקש‫<ref group=III>תוסיף: Mo30 שתוסיף; V397 שאוסיף<br> |
| + | מרובע: V397 המחובר marg. המרובע<br> | ||
| + | שהוא: Mo30 הוא<br> | ||
| + | בעצמו: Mo30; V397 בעצמו סוף החשבון<br> | ||
| + | כמה: Mo30 מה שיגיע<br> | ||
| + | וראה כמה המחובר: V397 om.</ref> | ||
|- | |- | ||
| | | | ||
*Example: we know that the square of 11 is 121, then we add to it 11, which is the root and it is the last number, the result is 132 and its half is 66. | *Example: we know that the square of 11 is 121, then we add to it 11, which is the root and it is the last number, the result is 132 and its half is 66. | ||
:<math>\scriptstyle{\color{blue}{\sum_{i=1}^{11} i=\frac{1}{2}\sdot\left(11^2+11\right)=\frac{1}{2}\sdot\left(121+11\right)=\frac{1}{2}\sdot132=66}}</math> | :<math>\scriptstyle{\color{blue}{\sum_{i=1}^{11} i=\frac{1}{2}\sdot\left(11^2+11\right)=\frac{1}{2}\sdot\left(121+11\right)=\frac{1}{2}\sdot132=66}}</math> | ||
| − | |style="text-align:right;"|<big>דמיון</big> ידענו כי מרובע י"א קכ"א ואחר<ref group=III>ואחר: Mo30 om.; V397 ואחר כן< | + | |style="text-align:right;"|<big>דמיון</big> ידענו כי מרובע י"א קכ"א ואחר נוסיף על י"א שהוא השרש והוא סוף החשבון יעלו קל"ב וחציו ס"ו‫<ref group=III>ואחר: Mo30 om.; V397 ואחר כן<br> |
| + | על: Mo30; V397 עליו<br> | ||
| + | השרש: Mo30 שרש</ref> | ||
|- | |- | ||
|From this way you can derive all the questions that are concerning this matter. | |From this way you can derive all the questions that are concerning this matter. | ||
| − | |style="text-align:right;"|ומזה הדרך | + | |style="text-align:right;"|ומזה הדרך תוכל להוציא כל השאלות שהם בענין הזה‫<ref group=III>הדרך: V397 om.<br> |
| + | הזה: Mo30 זה</ref> | ||
|- | |- | ||
| | | | ||
*{{#annot:aₙ, 1, 465|669|GXqn}}Example: one asks: I sum all the numbers until they reach a known number and the sum is 465. How much is the last number? | *{{#annot:aₙ, 1, 465|669|GXqn}}Example: one asks: I sum all the numbers until they reach a known number and the sum is 465. How much is the last number? | ||
:<math>\scriptstyle\sum_{i=1}^{n} i=465</math> | :<math>\scriptstyle\sum_{i=1}^{n} i=465</math> | ||
| − | |style="text-align:right;"|<big>דמיון</big> שאל שואל חברתי<ref group=III>חברתי: Mo30 חברנו< | + | |style="text-align:right;"|<big>דמיון</big> שאל שואל חברתי מספרים עד שהגיעו למספר ידוע ועלה המחובר תס"ה כמה הוא סוף החשבון{{#annotend:GXqn}}‫<ref group=III>חברתי: Mo30 חברנו<br> |
| + | מספרים: Mo30 כל המספרים; V397 המספרים<br> | ||
| + | שהגיעו: V397 שהגיע</ref> | ||
|- | |- | ||
|Always double the sum, then take the root of the preceding square and check it: if between the square and the double remains the root no more and no less, know that the calculation is correct and the [last] number is itself the root. | |Always double the sum, then take the root of the preceding square and check it: if between the square and the double remains the root no more and no less, know that the calculation is correct and the [last] number is itself the root. | ||
:<math>\scriptstyle\sum_{i=1}^{n} i=a\longrightarrow n=2a-n^2</math> | :<math>\scriptstyle\sum_{i=1}^{n} i=a\longrightarrow n=2a-n^2</math> | ||
| − | |style="text-align:right;"|כפול לעולם החשבון | + | |style="text-align:right;"|כפול לעולם החשבון המחובר וקח שרש הנכפל שעבר ובחון אותו כי אם נשאר בין המרובע ובין הנכפל כמו השרש בלי תוספת ומגרעת תדע כי החשבון נכון והחשבון בעצמו השרש‫<ref group=III>החשבון: Mo30; V397 חשבון<br> |
| + | ובחון: Mo30 ובחן<br> | ||
| + | השרש: Mo30; V397 הוא השרש</ref> | ||
|- | |- | ||
| | | | ||
:Hence, we double 465 and the result is 930. It is known that the preceding square is 900 and its root is 30, so it is the last summed number, for between the square and the double there is none but 30, thus it is the last number. | :Hence, we double 465 and the result is 930. It is known that the preceding square is 900 and its root is 30, so it is the last summed number, for between the square and the double there is none but 30, thus it is the last number. | ||
:<math>\scriptstyle{\color{blue}{n=\left(2\sdot465\right)-30^2=930-900=30}}</math> | :<math>\scriptstyle{\color{blue}{n=\left(2\sdot465\right)-30^2=930-900=30}}</math> | ||
| − | |style="text-align:right;"|והנה כפלנו | + | |style="text-align:right;"|והנה כפלנו תס"ה ועלה תתק"ל וידוע כי המרובע שעבר היה תת"ק ושרשו ל' והוא סוף המספרים המחוברים והנה אין בין המרובע והנכפל כי אם ל' והוא סוף החשבון‫<ref group=III>כפלנו: Mo30 כפל<br> |
| + | ועלה: Mo30 הוא; V397 ועלו<br> | ||
| + | וידוע: Mo30 וידענו<br> | ||
| + | היה: Mo30 om.; V397 היו<br> | ||
| + | המספרים ... סוף: Mo30 twice</ref> | ||
|- | |- | ||
|Another general way for an even and an odd number [of terms]: double the square of half the [last] number, then add to it the root of this square, which is half the number and the result is the required. | |Another general way for an even and an odd number [of terms]: double the square of half the [last] number, then add to it the root of this square, which is half the number and the result is the required. | ||
| Line 2,305: | Line 3,969: | ||
|Another question: we sum the squares up to a known number, how much is the sum? | |Another question: we sum the squares up to a known number, how much is the sum? | ||
:<math>\scriptstyle\sum_{i=1}^{n} i^2</math> | :<math>\scriptstyle\sum_{i=1}^{n} i^2</math> | ||
| − | |style="text-align:right;"|<big>שאלה אחרת</big> חברנו כל המרובעים<ref group=III>המרובעים: Mo30 המספרים marg. המרובעים< | + | |style="text-align:right;"|<big>שאלה אחרת</big> חברנו כל המרובעים שהם עד סוף החשבון שהוא ידוע כמה המחובר‫<ref group=III>המרובעים: Mo30 המספרים marg. המרובעים<br> |
| + | החשבון: Mo30 מספר<br> | ||
| + | שהוא: Mo30 om.</ref> | ||
|- | |- | ||
|You should know the sum of the numbers preceding the [last] mentioned number including [the last number] and we name it and call it a sum, then we take two thirds of the [last] mentioned number plus one third, multiply it by the sum and the result is the required, which is the sum of the squares up to the mentioned number. | |You should know the sum of the numbers preceding the [last] mentioned number including [the last number] and we name it and call it a sum, then we take two thirds of the [last] mentioned number plus one third, multiply it by the sum and the result is the required, which is the sum of the squares up to the mentioned number. | ||
:<math>\scriptstyle\sum_{i=1}^{n} i^2=\left(\sum_{i=1}^{n} i\right)\sdot\left[\left(\frac{2}{3}\sdot n\right)+\frac{1}{3}\right]</math> | :<math>\scriptstyle\sum_{i=1}^{n} i^2=\left(\sum_{i=1}^{n} i\right)\sdot\left[\left(\frac{2}{3}\sdot n\right)+\frac{1}{3}\right]</math> | ||
| − | |style="text-align:right;"|יש לך לדעת אותו החשבון שהזכיר כמה יעלה המחובר מהמספרים שלפניו ועמו נשים<ref group=III>נשים: Mo30 תשים< | + | |style="text-align:right;"|יש לך לדעת אותו החשבון שהזכיר כמה יעלה המחובר מהמספרים שלפניו ועמו נשים שם לאותו המספר הידוע ונקראנו סכום והנה נקח שתי שלישיות המספר שהזכיר עם תוספת שלישית אחד ונכפול זה המחובר על סכום המספר והעולה הוא המבוקש והוא המחובר מהמרובעים עד סוף המספר הנזכר‫<ref group=III>נשים: Mo30 תשים<br> |
| + | שם: V397 om.<br> | ||
| + | המספר: V397 מספר<br> | ||
| + | ונקראנו: Mo30 ותקראהו<br> | ||
| + | שלישית אחד: V397 שלישית אחד marg. כי הלויתי מהאחד<br> | ||
| + | ונכפול: Mo30 וכפול<br> | ||
| + | המחובר: Mo30 המספר</ref> | ||
|- | |- | ||
| | | | ||
*{{#annot:1-7|672|ad1Q}}Example: we wish to know how much are the squares up to seven? | *{{#annot:1-7|672|ad1Q}}Example: we wish to know how much are the squares up to seven? | ||
:<math>\scriptstyle\sum_{i=1}^{7} i^2</math> | :<math>\scriptstyle\sum_{i=1}^{7} i^2</math> | ||
| − | |style="text-align:right;"|<big>דמיון</big> רצינו לדעת כמה המרובעים שהם<ref group=III>המרובעים שהם: V397 הם המרובעים< | + | |style="text-align:right;"|<big>דמיון</big> רצינו לדעת כמה המרובעים שהם עד סוף שבעה{{#annotend:ad1Q}}‫<ref group=III>המרובעים שהם: V397 הם המרובעים<br> |
| + | שבעה: Mo30 שבעה והוא מספר שאין לו שלישית</ref> | ||
|- | |- | ||
| | | | ||
:We already know that the sum is 28, thus we turn to know how much are two thirds of a seven plus one third - they are five, for two thirds of a six are four and we have two thirds more plus one third, so we multiply the sum by 5 and the result is 140 which is the required. | :We already know that the sum is 28, thus we turn to know how much are two thirds of a seven plus one third - they are five, for two thirds of a six are four and we have two thirds more plus one third, so we multiply the sum by 5 and the result is 140 which is the required. | ||
| − | |style="text-align:right;"|וכבר<ref group=III>וכבר: Mo30 כבר< | + | |style="text-align:right;"|וכבר ידענו שהמחובר כ"ח ונשוב לדעת כמה שתי שלישיות שבעה עם תוספת שלישית אחד והם חמשה כי שתי שלישיות ששה הם ארבעה ויש לנו עוד שתי שלישיות ועם תוספת שלישית אחד והנה נכפול על הסכום ה' ועלו ק"מ והוא המבוקש‫<ref group=III>וכבר: Mo30 כבר<br> |
| + | שהמחובר: Mo30 כי המחובר<br> | ||
| + | ונשוב: V397 תשוב marg. ונשוב<br> | ||
| + | שבעה: V397 שלז‫'<br> | ||
| + | אחד: Mo30; V397 om.<br> | ||
| + | הם: V397 om.<br> | ||
| + | ויש: V397 יש<br> | ||
| + | אחד: Mo30 יהיה אחד<br> | ||
| + | והנה נכפול: Mo30 ונכפול</ref> | ||
|- | |- | ||
| colspan=2| | | colspan=2| | ||
| Line 2,326: | Line 4,007: | ||
*{{#annot:1-12|672|nrny}}Another example: how much is the sum of the squares up to 12? | *{{#annot:1-12|672|nrny}}Another example: how much is the sum of the squares up to 12? | ||
:<math>\scriptstyle\sum_{i=1}^{12} i^2</math> | :<math>\scriptstyle\sum_{i=1}^{12} i^2</math> | ||
| − | |style="text-align:right;"|<big>דמיון אחר</big> כמה המחובר<ref group=III>המחובר: Mo30 הוא המחובר< | + | |style="text-align:right;"|<big>דמיון אחר</big> כמה המחובר מהמרובעים שהם עד סוף י"ב{{#annotend:nrny}}‫<ref group=III>המחובר: Mo30 הוא המחובר<br> |
| + | שהם: Mo30 שהוא<br> | ||
| + | עד סוף: Mo30 מספר<br> | ||
| + | י"ב: Mo30 י"ב והוא מספר שיש לו שלישית</ref> | ||
|- | |- | ||
| | | | ||
:It is known that its sum is 78 and its two thirds are 8, we multiply it by 78, the result is 624, we add to it a third of the sum, which is 26, the result is 650 and it is the required. | :It is known that its sum is 78 and its two thirds are 8, we multiply it by 78, the result is 624, we add to it a third of the sum, which is 26, the result is 650 and it is the required. | ||
| − | |style="text-align:right;"|וידוע | + | |style="text-align:right;"|וידוע כי הסכום שלו ע"ח ושתי שלישיותיו ח' נכפלנו על ע"ח יעלו תרכ"ד נחבר אליו שלישית הסכום שהוא כ"ו יעלו תר"נ והוא המבוקש‫<ref group=III>וידוע: Mo30 וידענו<br> |
| + | יעלו: Mo30 ועלה<br> | ||
| + | נחבר: Mo30 ונחבר</ref> | ||
|- | |- | ||
| colspan=2| | | colspan=2| | ||
| Line 2,336: | Line 4,022: | ||
|- | |- | ||
|From this way you can derive all the questions that are concerning this matter. | |From this way you can derive all the questions that are concerning this matter. | ||
| − | |style="text-align:right;"|ועל זה הדרך תוכל להוציא כל השאלות שהם<ref group=III>שהם: V397 om.</ref> | + | |style="text-align:right;"|ועל זה הדרך תוכל להוציא כל השאלות שהם מזה הענין‫<ref group=III>שהם: V397 om.</ref> |
|- | |- | ||
!Additional excerpts | !Additional excerpts | ||
| Line 2,342: | Line 4,028: | ||
|- | |- | ||
|I have found an easy way to sum the cubes: | |I have found an easy way to sum the cubes: | ||
| − | |style="text-align:right;"|ואני<ref group=III>ואני: V397 om.< | + | |style="text-align:right;"|ואני מצאתי דרך נקלה לחבור המעוקבים‫<ref group=III>ואני: V397 om.<br> |
| + | לחבור: V397 לדעת</ref> | ||
|- | |- | ||
|If you want to know the [sum of the] cubes up to a known number, know the sum of the numbers up to that last number and take its square - it is the sum of the cubes up to the last number. | |If you want to know the [sum of the] cubes up to a known number, know the sum of the numbers up to that last number and take its square - it is the sum of the cubes up to the last number. | ||
:<math>\scriptstyle\sum_{i=1}^{n} i^3=\left(\sum_{i=1}^{n} i\right)^2</math> | :<math>\scriptstyle\sum_{i=1}^{n} i^3=\left(\sum_{i=1}^{n} i\right)^2</math> | ||
| − | |style="text-align:right;"|שאם תרצה לידע המעוקבים שהם עד מספר ידוע דע | + | |style="text-align:right;"|שאם תרצה לידע המעוקבים שהם עד מספר ידוע דע המספר המחובר עד סוף המספר ההוא וקח מרובעו והוא יהיה חבור המעוקבים עד סוף החשבון ההוא‫<ref group=III>שאם ... דע: V397 illegible<br> |
| + | מצאתי ... ההוא: V397 marg</ref> | ||
|- | |- | ||
| | | | ||
| Line 2,356: | Line 4,044: | ||
:The sum up to 5 is 15, take its square, it is 225 and it is the [sum] of the cubes up to 5. | :The sum up to 5 is 15, take its square, it is 225 and it is the [sum] of the cubes up to 5. | ||
:<math>\scriptstyle{\color{blue}{\sum_{i=1}^{5} i^3=\left(\sum_{i=1}^{5} i\right)^2=15^2=225}}</math> | :<math>\scriptstyle{\color{blue}{\sum_{i=1}^{5} i^3=\left(\sum_{i=1}^{5} i\right)^2=15^2=225}}</math> | ||
| − | |style="text-align:right;"|והנה מספר המחובר עד סוף ה' הוא ט"ו קח מרובעו יהיו רכ"ה והוא יהיה מספר המעוקבים עד ה‫'<ref group=III>דמיון ... ה': V397 om.</ref> | + | |style="text-align:right;"|והנה מספר המחובר עד סוף ה' הוא ט"ו קח מרובעו יהיו רכ"ה והוא יהיה מספר המעוקבים עד ה‫'<ref group=III>דמיון ... ה‫': V397 om.</ref> |
|- | |- | ||
|Another matter: to know the sum of all the doubles. | |Another matter: to know the sum of all the doubles. | ||
:<math>\scriptstyle\sum_{i=1}^{n} \left(a_1\sdot2^{i-1}\right)</math> | :<math>\scriptstyle\sum_{i=1}^{n} \left(a_1\sdot2^{i-1}\right)</math> | ||
| − | |style="text-align:right;"|‫<ref group=III>Mo30; V397 om.</ref> | + | |style="text-align:right;"|<big>ענין אחר</big> לדעת כמה המחובר מכל הנכפל‫<ref group=III>Mo30; V397 om.</ref> |
|- | |- | ||
|It is [equal to] double the last minus the first. | |It is [equal to] double the last minus the first. | ||
| Line 2,379: | Line 4,067: | ||
*Another example: 1, 2, 4 | *Another example: 1, 2, 4 | ||
:<math>\scriptstyle\sum_{i=1}^{3} \left(1\sdot2^{i-1}\right)</math> | :<math>\scriptstyle\sum_{i=1}^{3} \left(1\sdot2^{i-1}\right)</math> | ||
| − | |style="text-align:right;"|<big>דמיון אחר</big> א' ב' ד' | + | |style="text-align:right;"|<big>דמיון אחר</big> א' ב' ד‫' |
|- | |- | ||
| | | | ||
| Line 2,433: | Line 4,121: | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|ועל הדרך הזה | + | |style="text-align:right;"|ועל הדרך הזה תוכל להוציא כל השאלות מזה הענין‫<ref group=III>הדרך הזה: Mo30 זה הדרך<br> |
| + | כל השאלות מזה הענין: Mo30 השאר<br>ועל הדרך ... מזה הענין: V397 om.</ref> | ||
|- | |- | ||
|There is no need to mention all of this, but I shall only mention the method of the scales. | |There is no need to mention all of this, but I shall only mention the method of the scales. | ||
| − | |style="text-align:right;"|ואין צורך להזכיר כל<ref group=III>כל: V397 om.< | + | |style="text-align:right;"|ואין צורך להזכיר כל זה רק אזכיר דרך המאזנים‫<ref group=III>כל: V397 om.<br> |
| + | כל זה: Mo30 om.</ref> | ||
|- | |- | ||
| | | | ||
| Line 2,445: | Line 4,135: | ||
|- | |- | ||
|When you add a number to a number, even if they [consist of] many [digits], put one number in the upper row according to its ranks, put also the other number according to its ranks in the lower row. | |When you add a number to a number, even if they [consist of] many [digits], put one number in the upper row according to its ranks, put also the other number according to its ranks in the lower row. | ||
| − | |style="text-align:right;"|כש{{#annot:term|178|vIio}}תחבר | + | |style="text-align:right;"|כש{{#annot:term|178|vIio}}תחבר מספר אל מספר{{#annotend:vIio}} בין שיהיו רבים אלו ואלו שים המספר האחד בטור העליון כפי מעלותיו גם שים המספר השני כפי מעלותיו בטור השפל‫<ref group=III>כשתחבר: V397 בשחבר<br> |
| + | שיהיו: Mo30 שיהיה<br> | ||
| + | אלו ואלו: V397 אלה על אלה<br> | ||
| + | האחד: V397 om.<br> | ||
| + | העליון: V397 אחד<br> | ||
| + | מעלותיו: V397 מעלתו<br> | ||
| + | גם: V397 וגם<br> | ||
| + | המספר: V397 מספר<br> | ||
| + | מעלותיו: V397 מעלתו</ref> | ||
|- | |- | ||
|Then add each [digit] in its rank and write the sum in a third row. | |Then add each [digit] in its rank and write the sum in a third row. | ||
| Line 2,451: | Line 4,149: | ||
|- | |- | ||
|Then add the scale of the top row to the scale of the bottom row. | |Then add the scale of the top row to the scale of the bottom row. | ||
| − | |style="text-align:right;"|אחר כן חבר מאזני הטור העליון אל | + | |style="text-align:right;"|אחר כן חבר מאזני הטור העליון אל מאזני הטור התחתון‫<ref group=III>אל: V397 עם<br> |
| + | התחתון: V397 השפל</ref> | ||
|- | |- | ||
|If the sum of the two is equal to the scale of the third row, know that your calculation is correct. | |If the sum of the two is equal to the scale of the third row, know that your calculation is correct. | ||
| − | |style="text-align:right;"|ואם היה העולה בין שניהם כמאזני הטור השלישי<ref group=III>השלישי: V397 השפל</ref> | + | |style="text-align:right;"|ואם היה העולה בין שניהם כמאזני הטור השלישי אז תדע כי חשבונך אמת‫<ref group=III>השלישי: V397 השפל</ref> |
|- | |- | ||
|Now I shall explain how to approach the tables of astrology and how to take add seconds to minutes and minutes to degrees and degrees to signs. | |Now I shall explain how to approach the tables of astrology and how to take add seconds to minutes and minutes to degrees and degrees to signs. | ||
| Line 2,474: | Line 4,173: | ||
|- | |- | ||
|Each minute is also divided into sixtieths, which are called seconds. | |Each minute is also divided into sixtieths, which are called seconds. | ||
| − | |style="text-align:right;"|גם כל<ref group=III>כל: V397 על< | + | |style="text-align:right;"|גם כל ראשון יתחלק לששים עוד ויקראו שניים‫<ref group=III>כל: V397 על<br> |
| + | עוד: V397 ועוד</ref> | ||
|- | |- | ||
|There are no more fractions than these in the tables of the planets. | |There are no more fractions than these in the tables of the planets. | ||
| Line 2,484: | Line 4,184: | ||
| | | | ||
*One according to the solar years, in which the years of the decades are summed twenty by twenty. | *One according to the solar years, in which the years of the decades are summed twenty by twenty. | ||
| − | |style="text-align:right;"|האחד על שנות השמש והם שנות הכלל מחוברים עשרים עשרים‫<ref group=III>עשרים עשרים: V397 כ' על כ'</ref> | + | |style="text-align:right;"|האחד על שנות השמש והם שנות הכלל מחוברים עשרים עשרים‫<ref group=III>עשרים עשרים: V397 כ' על כ‫'</ref> |
|- | |- | ||
| | | | ||
| Line 2,491: | Line 4,191: | ||
|- | |- | ||
|I will explain this in the book | |I will explain this in the book | ||
| − | |style="text-align:right;"|ובספר<ref group=III>ובספר: V397 ובמספר</ref> | + | |style="text-align:right;"|ובספר טעמי הלוחות אפרש זה‫<ref group=III>ובספר: V397 ובמספר</ref> |
|- | |- | ||
|When they want to know the position of any planet required at any hour required, they approach the years of the decades that have passed and write down the number of the signs they find written there, and they write this at the beginning of a row. | |When they want to know the position of any planet required at any hour required, they approach the years of the decades that have passed and write down the number of the signs they find written there, and they write this at the beginning of a row. | ||
| − | |style="text-align:right;"|והנה ברצותם לדעת | + | |style="text-align:right;"|והנה ברצותם לדעת מקום איזה משרת שיבקשו באיזו שעה שירצו יכנסו בשנות הכלל שעברו ויכתבו מה שימצאו כתוב שם ממספר המזלות ויכתבו זה בתחלת הטור‫<ref group=III>לדעת: V397 om.<br> |
| + | שיבקשו: V397 יבקשו<br> | ||
| + | זה: V397 שם <sup>זה</sup></ref> | ||
|- | |- | ||
|Then, they write the degrees they find, after the first number in the same row and they do a long dividing line between the two numbers. | |Then, they write the degrees they find, after the first number in the same row and they do a long dividing line between the two numbers. | ||
| Line 2,500: | Line 4,202: | ||
|- | |- | ||
|Then, they write the minutes they want find after the degrees and the signs and make a separation between the two numbers with a long line in the same row. | |Then, they write the minutes they want find after the degrees and the signs and make a separation between the two numbers with a long line in the same row. | ||
| − | |style="text-align:right;"|אחר כן יכתבו מה שימצאו מן הראשונים | + | |style="text-align:right;"|אחר כן יכתבו מה שימצאו מן הראשונים אחרי המעלות והמזלות וישימו הפרש בין שני המספרים בקו ארוך באותו הטור בעצמו‫<ref group=III>מן הראשונים: V397 מהראשונים<br> |
| + | באותו: V397 <s>המעלות והמזלות ויש</s> באותו</ref> | ||
|- | |- | ||
|Then, they write the seconds after the minutes in the same row and separate between them with a long line. | |Then, they write the seconds after the minutes in the same row and separate between them with a long line. | ||
| Line 2,506: | Line 4,209: | ||
|- | |- | ||
|Then, they approach the single years that have passed and write what they find, the signs under the signs, the degrees under the degrees, the minutes under the minutes and the seconds under the seconds. | |Then, they approach the single years that have passed and write what they find, the signs under the signs, the degrees under the degrees, the minutes under the minutes and the seconds under the seconds. | ||
| − | |style="text-align:right;"|ואחר כן יכנסו בשנות הפרט שעברו ויכתבו מה שימצאו<ref group=III>שימצאו: V397 שנמצאות</ref> | + | |style="text-align:right;"|ואחר כן יכנסו בשנות הפרט שעברו ויכתבו מה שימצאו במזלות תחת המזלות והמעלות תחת המעלות והראשונים תחת הראשונים והשניים תחת השניים‫<ref group=III>שימצאו: V397 שנמצאות</ref> |
|- | |- | ||
|Then, they approach the months that have passed and write everything they find there, each type under its type. | |Then, they approach the months that have passed and write everything they find there, each type under its type. | ||
| Line 2,518: | Line 4,221: | ||
|- | |- | ||
|Then, one starts to add up all the sixtieths: takes one minute for every sixty seconds and write how many minutes come out of the seconds with the minutes that precede the seconds, and write the remainder of the seconds that are less than sixty alone in another place. | |Then, one starts to add up all the sixtieths: takes one minute for every sixty seconds and write how many minutes come out of the seconds with the minutes that precede the seconds, and write the remainder of the seconds that are less than sixty alone in another place. | ||
| − | |style="text-align:right;"|ואחר כן יחל לחבר כל הששים ויקח לכל ששים שניים חלק ראשון ויכתוב כמה ראשונים יעלו מן השניים עם הראשונים שהם לפני השניים והנשאר מן השניים שהם פחותים מן הששים<ref group=III>מן הששים: V397 משישים< | + | |style="text-align:right;"|ואחר כן יחל לחבר כל הששים ויקח לכל ששים שניים חלק ראשון ויכתוב כמה ראשונים יעלו מן השניים עם הראשונים שהם לפני השניים והנשאר מן השניים שהם פחותים מן הששים יכתבם לבדד במקום אחר‫<ref group=III>מן הששים: V397 משישים<br> |
| + | במקום: V397 במקום <s>במקום</s></ref> | ||
|- | |- | ||
|Then, one adds again all the minutes: takes one degree for every sixty minutes and write the degrees that are summed with the degrees that precede the minutes and the remaining minutes that are less than sixty, write them in the row where you wrote the seconds, only that they are written before the seconds. | |Then, one adds again all the minutes: takes one degree for every sixty minutes and write the degrees that are summed with the degrees that precede the minutes and the remaining minutes that are less than sixty, write them in the row where you wrote the seconds, only that they are written before the seconds. | ||
| − | |style="text-align:right;"|ואחר כן ישוב לחבר כל הראשונים ויקח לכל ששים ראשונים מעלה אחת ומה שיתחבר מן המעלות | + | |style="text-align:right;"|ואחר כן ישוב לחבר כל הראשונים ויקח לכל ששים ראשונים מעלה אחת ומה שיתחבר מן המעלות כתוב אותם עם המעלות שהיו לפני הראשונים והראשונים הנשארים שהם פחותים מששים כתוב אותם בטור שכתבת שם השניים רק יהיו נכתבים לפני השניים‫<ref group=III>מן המעלות: V397 מהמעלות<br> |
| + | שכתבת: V397 <s>כמו</s> שכתבת</ref> | ||
|- | |- | ||
|Then, add again the degrees: take one sign for every thirty degrees and write the [signs] that come out with all the [signs] that you had and what remains of the degrees that are less than thirty, write them alone before the minutes that you wrote before the seconds. | |Then, add again the degrees: take one sign for every thirty degrees and write the [signs] that come out with all the [signs] that you had and what remains of the degrees that are less than thirty, write them alone before the minutes that you wrote before the seconds. | ||
| − | |style="text-align:right;"|ואחר כן שוב לחבר המעלות וקח לכל שלשים מעלות מזל אחד וכתוב המעלות<ref group=III>המעלות: V397 <s>המעלות</s> marg. המזלות< | + | |style="text-align:right;"|ואחר כן שוב לחבר המעלות וקח לכל שלשים מעלות מזל אחד וכתוב המעלות העולים עם כל המעלות שהיו לך ומה שישארו מן המעלות שהם פחותים משלשים כתוב אותם לפני הראשונים שכתבת לפני השניים לבדד‫<ref group=III>המעלות: V397 <s>המעלות</s> marg. המזלות<br> |
| + | המעלות: V397 המזלות<br> | ||
| + | מן המעלות: V397 מהמעלות</ref> | ||
|- | |- | ||
|Then, take out every twelve signs you find, and write the remainder alone before the degrees you wrote before the minutes preceding the seconds. | |Then, take out every twelve signs you find, and write the remainder alone before the degrees you wrote before the minutes preceding the seconds. | ||
| − | |style="text-align:right;"|ואחר כן הוצא כל שנים עשר מזלות<ref group=III>מזלות: V397 om.</ref> | + | |style="text-align:right;"|ואחר כן הוצא כל שנים עשר מזלות שתמצא והנשאר כתוב אותם לפני המעלות שכתבת לפני הראשונים שכתבת לפני השניים לבדד‫<ref group=III>מזלות: V397 om.</ref> |
|- | |- | ||
|So, you have the position of the planet on the celestial sphere along with its degrees, minutes and seconds. | |So, you have the position of the planet on the celestial sphere along with its degrees, minutes and seconds. | ||
| − | |style="text-align:right;"|אז<ref group=III>אז: V397 <s>אז</s> marg. אז</ref> | + | |style="text-align:right;"|אז יהיה לך מקום המשרת בגלגל המזלות עם מעלותיו וחלקיו ושנייו‫<ref group=III>אז: V397 <s>אז</s> marg. אז</ref> |
|- | |- | ||
|You always start to count the signs from the sign of Aries. | |You always start to count the signs from the sign of Aries. | ||
| Line 2,536: | Line 4,243: | ||
|- | |- | ||
|If the number of signs is twelve, write a zero in the place where the signs must be written to indicate that the planet is still in the sign of Aries, for this is not yet complete, but that it has only moved away from the sign in accordance with the degrees that have been written there and the minutes belong to the following degree. | |If the number of signs is twelve, write a zero in the place where the signs must be written to indicate that the planet is still in the sign of Aries, for this is not yet complete, but that it has only moved away from the sign in accordance with the degrees that have been written there and the minutes belong to the following degree. | ||
| − | |style="text-align:right;"|ואם היה מספר המזלות שנים עשר כתוב אותם במקום הראוי להכתב שם | + | |style="text-align:right;"|ואם היה מספר המזלות שנים עשר כתוב אותם במקום הראוי להכתב שם מזלות גלגל להודיע כי המשרת עודנו במזל טלה כי לא נשלם רק עבר מן המזל כפי המעלות שתמצא כתובות ויהיו הראשונים מן המעלה הבאה‫<ref group=III>שם: V397 om.<br> |
| + | מן המעלה: V397 מהמעלה</ref> | ||
|- | |- | ||
| | | | ||
::For, if the degrees are 17, the minutes are those that have passed from the 18th degree. Suppose the whole minutes are 15 and say the seconds are 45, so they are three-quarters of one minute of sixteen minutes. | ::For, if the degrees are 17, the minutes are those that have passed from the 18th degree. Suppose the whole minutes are 15 and say the seconds are 45, so they are three-quarters of one minute of sixteen minutes. | ||
| − | |style="text-align:right;"|כי אם | + | |style="text-align:right;"|כי אם היו המעלות י"ז יהיו הראשונים הם שעברו ממעלת י"ח ונחשוב כי הראשונים השלמים היו ט"ו שלמים ונאמר כי השניים היו מ"ה והנה הם שלש רביעיות החלק הראשון של ששה עשר ראשונים‫<ref group=III>כי אם: V397 <s>כי אם</s> כי אם<br> |
| + | ממעלת: V397 ממעלות</ref> | ||
|- | |- | ||
| | | | ||
| Line 2,557: | Line 4,266: | ||
|- | |- | ||
|I shall give you only a general method of subtracting many digits from many digits, using the method of the arithmeticians as well as the method of the astrologers, for they have a different method. | |I shall give you only a general method of subtracting many digits from many digits, using the method of the arithmeticians as well as the method of the astrologers, for they have a different method. | ||
| − | |style="text-align:right;"|רק אתן לך דרך כוללת לגרוע חשבונים רבים מחשבונים רבים | + | |style="text-align:right;"|רק אתן לך דרך כוללת לגרוע חשבונים רבים מחשבונים רבים על דרך חכמי החשבון גם על דרך חכמי המזלות כי דרך אחרת יש להם‫<ref group=IV>מחשבונים רבים: V397 marg.<br> |
| + | אחרת: V397 אמת</ref> | ||
|- | |- | ||
|Do as follows: | |Do as follows: | ||
| Line 2,563: | Line 4,273: | ||
|- | |- | ||
|Write the number from which you want to subtract [= the minuend] in the upper row and write the digits you want to subtract [= the subtrahend] in the lower row. | |Write the number from which you want to subtract [= the minuend] in the upper row and write the digits you want to subtract [= the subtrahend] in the lower row. | ||
| − | |style="text-align:right;"|כתוב החשבון | + | |style="text-align:right;"|כתוב החשבון שתרצה לגרוע ממנו בטור העליון וכתוב המספרים שתחפוץ לגרעם בטור השפל‫<ref group=IV>החשבון: V397 המספר<br> |
| + | שתחפוץ: V397 שתרצה<br> | ||
| + | לגרעם: V397 לגרוע אותם</ref> | ||
|- | |- | ||
|The last digit in the top row must always be greater than the last digit in the bottom row. Do not consider the other digits. | |The last digit in the top row must always be greater than the last digit in the bottom row. Do not consider the other digits. | ||
| Line 2,569: | Line 4,281: | ||
|- | |- | ||
|If you find in one of the ranks that the digit in the lower row is greater than the corresponding digit in the upper row, return one alone from the digit that follows, for that is enough for you and consider it as ten, as the way we do in the division. | |If you find in one of the ranks that the digit in the lower row is greater than the corresponding digit in the upper row, return one alone from the digit that follows, for that is enough for you and consider it as ten, as the way we do in the division. | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<div id="if you find in one of the ranks"></div>והנה אם מצאת באחת מן המעלות‫<ref group=note>[[פירוש_אנונימי_לספר_המספר#If_you_find_in_one_of_the_ranks|<span style=color:blue>commentary: if you find in one of the ranks</span>]]</ref> מספר הטור השפל גדול מהמספר שבטור העליון שהוא כנגדו השב אחורנית מהמספר שאחריו אחד לבדו כי יספיק לך וחשוב אותו עשרה על הדרך שאנו עושין בחלוק‫<ref group=IV>והנה: V397 וכן<br> |
| + | באחת: V397 באחדות<br> | ||
| + | מן: V397 om.<br> | ||
| + | מהמספר: V397 ממספר<br> | ||
| + | שבטור: V397 הטור<br> | ||
| + | שאחריו: V397 שהוא אחריו<br> | ||
| + | אחד: V397 ואחד</ref> | ||
|- | |- | ||
| | | | ||
| Line 2,578: | Line 4,296: | ||
| | | | ||
:Each [digit] of the bottom should be subtracted from the top [digits], each one from its rank: 2 from 5 and 3 from 4. | :Each [digit] of the bottom should be subtracted from the top [digits], each one from its rank: 2 from 5 and 3 from 4. | ||
| − | |style="text-align:right;"|וראוי לגרוע כל אחד מהשפלים מן העליונים<ref group=IV>מן העליונים: V397 מהעליונים< | + | |style="text-align:right;"|וראוי לגרוע כל אחד מהשפלים מן העליונים כל אחד ממעלתו ב' מה' וג' מד‫'‫<ref group=IV>מן העליונים: V397 מהעליונים<br> |
| + | ממעלתו: V397 ממעלותו</ref> | ||
|- | |- | ||
| | | | ||
:But we cannot subtract 7 from 3, nor 9 from 2. | :But we cannot subtract 7 from 3, nor 9 from 2. | ||
| − | |style="text-align:right;"|ולא נוכל לחסר ז' מג' ולא ט' מב‫'<ref group=IV>ולא נוכל ... מב': V397 om.</ref> | + | |style="text-align:right;"|ולא נוכל לחסר ז' מג' ולא ט' מב‫'<ref group=IV>ולא נוכל ... מב‫': V397 om.</ref> |
|- | |- | ||
|We always start to subtract backwards, as with the way of division, and write the remainder in the third row corresponding to the same rank in the lower row. | |We always start to subtract backwards, as with the way of division, and write the remainder in the third row corresponding to the same rank in the lower row. | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<div id="we always subtract backwards">ולעולם נחל לחסר אחורנית<ref>MS St.P374 excerpt 1 end</ref> כדרך החלוק</div>‫<ref group=note>[[Excerpts_Attributed_to_Immanuel_Bonfils#We_always_subtract_backwards|<span style=color:blue>commentary: we always subtract backwards</span>]]</ref> והנשאר נכתבנו בטור השלישי כנגד אותה המעלה שבטור השפל‫<ref group=IV>ולעולם: V397 וככה הכל ולעולם<br> |
| + | לחסר: V397 ולהסר<br> | ||
| + | נכתבנו: V397 נכתוב אותו</ref> | ||
|- | |- | ||
| | | | ||
:*So, we subtract 2 from 5; we are left with 3; we write it beneath the fourth rank. | :*So, we subtract 2 from 5; we are left with 3; we write it beneath the fourth rank. | ||
| − | |style="text-align:right;"|והנה גרענו ב' מה' נשאר לנו ג' כתבנו אותו<ref group=IV>כתבנו אותו: V397 כתבנוהו< | + | |style="text-align:right;"|והנה גרענו ב' מה' נשאר לנו ג' כתבנו אותו תחת מעלה הרביעית‫<ref group=IV>כתבנו אותו: V397 כתבנוהו<br> |
| + | תחת מעלה הרביעית: V397 om.</ref> | ||
|- | |- | ||
| | | | ||
:*We subtract 3 from 4; we are left with one, but we do not write it down, we only put a zero instead, because we have to return one back, for the preceding digit that is in the lower row is greater than the corresponding upper one. So, they are 13. We subtract 7 from it; 6 remain. However, because the first digit in the bottom row is greater than the [first digit] in the top row, we have to return one back and write 5 in the third row. So, there are 12 above; we subtract 9, 3 remain. | :*We subtract 3 from 4; we are left with one, but we do not write it down, we only put a zero instead, because we have to return one back, for the preceding digit that is in the lower row is greater than the corresponding upper one. So, they are 13. We subtract 7 from it; 6 remain. However, because the first digit in the bottom row is greater than the [first digit] in the top row, we have to return one back and write 5 in the third row. So, there are 12 above; we subtract 9, 3 remain. | ||
| − | |style="text-align:right;"|חסרנו ג' מד' נשאר לנו אחד ולא כתבנוהו רק שמנו גלגל במקומו<ref group=IV>שמנו גלגל במקומו: V397 כתבנו במקומו גלגל< | + | |style="text-align:right;"|חסרנו ג' מד' נשאר לנו אחד ולא כתבנוהו רק שמנו גלגל במקומו כי הוצרכנו להשיב אחד אחורנית כי המספר שבטור השפל לפניו גדול משכנגדו העליון והנה היו י"ג חסרנו ממנו ז' ונשארו ו' רק בעבור כי החשבון הראשון שבטור השפל גדול מן העליון שבטור העליון על כן הוצרכנו להשיב אחורנית אחד ונכתוב ה' בטור השלישי והנה היו למעלה י"ב נחסר ט' ונשארו ג‫'‫<ref group=IV>שמנו גלגל במקומו: V397 כתבנו במקומו גלגל<br> |
| + | אחד: V397 top<br> | ||
| + | שבטור השפל לפניו: V397 שהוא לפניו בטור השפל<br> | ||
| + | ונשארו: V397 נשאר<br> | ||
| + | כי: V397 שהוא<br> | ||
| + | שבטור השפל: V397 בשפל<br> | ||
| + | מן: V397 משל<br> | ||
| + | שבטור: V397 בטור<br> | ||
| + | ונכתוב: V397 וכתבנו<br> | ||
| + | היו: V397 היה לנו<br> | ||
| + | נחסר ט‫': V397 om.<br> | ||
| + | ונשארו: V397 נשארו</ref> | ||
|- | |- | ||
| | | | ||
| Line 2,600: | Line 4,333: | ||
|- | |- | ||
|When you want to know the scales: | |When you want to know the scales: | ||
| − | |style="text-align:right;"|וכאשר תרצה<ref group=IV>תרצה: V397 נרצה</ref> | + | |style="text-align:right;"|וכאשר תרצה לדעת המאזנים‫<ref group=IV>תרצה: V397 נרצה</ref> |
|- | |- | ||
|Subtract the scale of the lower row from the scales of the upper row and keep the remainder. | |Subtract the scale of the lower row from the scales of the upper row and keep the remainder. | ||
| − | |style="text-align:right;"|תגרע | + | |style="text-align:right;"|תגרע מאזני הטור השפל ממאזני הטור העליון ותשמור הנשאר‫<ref group=IV>תגרע: V397 נגרע<br> |
| + | ותשמור: V397 ונשמור</ref> | ||
|- | |- | ||
|See: if the scale of the third row is the same, then you know that your calculation is correct. | |See: if the scale of the third row is the same, then you know that your calculation is correct. | ||
| − | |style="text-align:right;"|ותראה<ref group=IV>ותראה: V397 ונראה< | + | |style="text-align:right;"|ותראה אם היו מאזני הטור השלישי כמוהו דע כי חשבונך אמת‫<ref group=IV>ותראה: V397 ונראה<br> |
| + | היו: V397 om.<br> | ||
| + | דע: V397 נדע<br> | ||
| + | חשבונך: V397 חשבוננו</ref> | ||
|- | |- | ||
|If the scale of the bottom row is greater than the scale of the top row, always add 9 to the scale of the top, subtract the scale of the bottom from the sum and proceed according to the instructions. | |If the scale of the bottom row is greater than the scale of the top row, always add 9 to the scale of the top, subtract the scale of the bottom from the sum and proceed according to the instructions. | ||
| − | |style="text-align:right;"|ואם היו<ref group=IV>היו: V397 היה< | + | |style="text-align:right;"|ואם היו מאזני הטור השפל גדול ממאזני הטור העליון הוסף לעולם על מאזני העליון ט' וגרע מהמחובר מאזני השפל ותעשה כמשפט‫<ref group=IV>היו: V397 היה<br> |
| + | הטור: V397 om.<br> | ||
| + | הוסף: V397 ה<sup>ו</sup>סף<br> | ||
| + | ותעשה: V397 ונעשה<br> | ||
| + | כמשפט: V397 <s>השפל</s> marg. כמשפט</ref> | ||
|- | |- | ||
|Example. We subtract 1 from 2; 1 remains. We return it back to the zero; they are 10. We subtract 7; 3 remain. | |Example. We subtract 1 from 2; 1 remains. We return it back to the zero; they are 10. We subtract 7; 3 remain. | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<big>דמיון</big> החסרנו א' מב' נשאר א' השיבונו אחורנית על הגלגל והיו י' חסרנו ז' נשארו ג‫'‫<ref group=IV>החסרנו: V397 חסרנו<br> |
| + | השיבונו: V397 השיבונו אותו<br> | ||
| + | והיו י' חסרנו ז‫': V397 om.</ref> | ||
|- | |- | ||
|The scale of the lower [row] is 8 and the scale of the upper [row] is 2. We add 9 to it; they are 11. We subtract 8; 3 remain and so is the bottom scale. | |The scale of the lower [row] is 8 and the scale of the upper [row] is 2. We add 9 to it; they are 11. We subtract 8; 3 remain and so is the bottom scale. | ||
| − | |style="text-align:right;"|והנה מאזני השפל ח' ומאזני העליון ב' הוספנו עליו ט' והיו<ref group=IV>והיו: V397 היו< | + | |style="text-align:right;"|והנה מאזני השפל ח' ומאזני העליון ב' הוספנו עליו ט' והיו י"א החסרנו ח' נשארו ג' וככה מאזני השפל‫<ref group=IV>והיו: V397 היו<br> |
| + | החסרנו: V397 חסרנו</ref> | ||
|- | |- | ||
|Now, we shall speak about the method of the astrologers, because they need this chapter more than the arithmeticians. | |Now, we shall speak about the method of the astrologers, because they need this chapter more than the arithmeticians. | ||
| − | |style="text-align:right;"|עתה נדבר על דרך חכמי<ref>MS N2624 end</ref> המזלות כי יותר צורך יש<ref group=IV>יש: V397 om.</ref> | + | |style="text-align:right;"|עתה נדבר על דרך חכמי‫<ref>MS N2624 end</ref> המזלות כי יותר צורך יש להם לשער הזה מחכמי החשבון‫<ref group=IV>יש: V397 om.</ref> |
|- | |- | ||
|We already mentioned that you should set up the signs in the first rank, degrees in the second, the minutes in the third and seconds in the fourth. | |We already mentioned that you should set up the signs in the first rank, degrees in the second, the minutes in the third and seconds in the fourth. | ||
| − | |style="text-align:right;"|וכבר הזכרנו כי כן תכון<ref group=IV>כן תכון: V397 om.< | + | |style="text-align:right;"|וכבר הזכרנו כי כן תכון המזלות במעלה הראשונה והמעלות בשנית והראשונים בשלישית<ref>MS St.P569 excerpt 1 end</ref> והשניים ברביעית‫<ref group=IV>כן תכון: V397 om.<br> |
| + | והראשונים: V397 והחלקים הראשונים</ref> | ||
|- | |- | ||
|One always starts to subtract backwards: the seconds in the bottom row from the seconds in the top row. The remainder is written in the third row corresponding to the upper seconds. | |One always starts to subtract backwards: the seconds in the bottom row from the seconds in the top row. The remainder is written in the third row corresponding to the upper seconds. | ||
| − | |style="text-align:right;"|ולעולם יחלו לגרוע אחורנית השניים<ref group=IV>השניים: V397 כי השניים< | + | |style="text-align:right;"|ולעולם יחלו לגרוע אחורנית השניים בטור השפל מהשניים בטור העליון והנשאר יכתבהו בטור השלישי כנגד השניים העליונים‫<ref group=IV>השניים: V397 כי השניים<br> |
| + | השפל: V397 שפל<br> | ||
| + | מהשניים: V397 יחלו לגרוע מהשניים<br> | ||
| + | יכתבהו: V397 יכתבנו</ref> | ||
|- | |- | ||
|If the lower seconds are more than the upper ones, he takes one from the upper minutes, considers it as 60 seconds, adds to them the seconds that are in the upper row, and then subtracts the lower seconds according to the instructions. | |If the lower seconds are more than the upper ones, he takes one from the upper minutes, considers it as 60 seconds, adds to them the seconds that are in the upper row, and then subtracts the lower seconds according to the instructions. | ||
| − | |style="text-align:right;"|ואם היו השניים השפלים רבים מהעליונים יקח מהראשונים העליונים<ref group=IV>העליונים: V397 om.< | + | |style="text-align:right;"|ואם היו השניים השפלים רבים מהעליונים יקח מהראשונים העליונים אחד יחשבהו ס' שניים ויחבר אליהם השניים הנמצאים בטור העליון ואחר כן יגרע השניים השפלים כמשפט‫<ref group=IV>העליונים: V397 om.<br> |
| + | יחשבהו: V397 ויחשוב אותו</ref> | ||
|- | |- | ||
|If he has taken one from the upper [minutes], he subtracts it from the number of minutes that are there. | |If he has taken one from the upper [minutes], he subtracts it from the number of minutes that are there. | ||
| Line 2,639: | Line 4,388: | ||
|- | |- | ||
|If the degrees in the lower row are more than the degrees in the upper row, he takes one from the signs, considers it as 30, adds them to the degrees that are written there, then subtracts, but makes sure that he subtracts one from the number of the signs that are written in the first [rank]. | |If the degrees in the lower row are more than the degrees in the upper row, he takes one from the signs, considers it as 30, adds them to the degrees that are written there, then subtracts, but makes sure that he subtracts one from the number of the signs that are written in the first [rank]. | ||
| − | |style="text-align:right;"|ואם היו המעלות בטור השפל רבות ממעלות הטור העליון יקח מהמזלות<ref group=IV>מהמזלות: V397 מהמעלות marg. מהמזלות< | + | |style="text-align:right;"|ואם היו המעלות בטור השפל רבות ממעלות הטור העליון יקח מהמזלות אחד ויחשבנו ל' ויחברם אל המעלות הכתובות שם ואחר כך יגרע וישמר שיגרע אחד ממספר המזלות הכתובים בראשונה‫<ref group=IV>מהמזלות: V397 מהמעלות marg. מהמזלות<br> |
| + | אחד: V397 om.<br> | ||
| + | ויחשבנו: V397 וי<s>ת</s><sup>ח</sup>שבנו<br> | ||
| + | ל‫': V397 למעלה marg. למעלות</ref> | ||
|- | |- | ||
|Then, he subtracts the signs from the signs and write the remainder in the third row corresponding to them. | |Then, he subtracts the signs from the signs and write the remainder in the third row corresponding to them. | ||
| − | |style="text-align:right;"|ואחר כך יגרע<ref group=IV>וישמר ... יגרע: V397 marg.</ref> | + | |style="text-align:right;"|ואחר כך יגרע מזלות ממזלות ויכתוב הנשארים בטור השלישי כנגדם‫<ref group=IV>וישמר ... יגרע: V397 marg.</ref> |
|- | |- | ||
|If the lower signs are more than the upper ones, he always adds 12 to the upper ones, then subtracts and proceeds according to the instructions. | |If the lower signs are more than the upper ones, he always adds 12 to the upper ones, then subtracts and proceeds according to the instructions. | ||
| − | |style="text-align:right;"|ואם היו המזלות השפלים גדולים<ref group=IV>גדולים: V397 הגדולים< | + | |style="text-align:right;"|ואם היו המזלות השפלים גדולים מהעליונים יוסיף לעולם על העליונים י"ב ואחר כן יגרע ויעשה כמשפט‫<ref group=IV>גדולים: V397 הגדולים<br> |
| + | יוסיף: V397 נוסיף<br> | ||
| + | לעולם: V397 om.</ref> | ||
|- | |- | ||
|To know the scales: | |To know the scales: | ||
| Line 2,651: | Line 4,405: | ||
|- | |- | ||
|One starts with the seconds, subtracts the scale of the lower seconds from the scale of the upper ones, keeps the remainder and see if the scale of seconds in the third row is just as much, then his calculation is correct. | |One starts with the seconds, subtracts the scale of the lower seconds from the scale of the upper ones, keeps the remainder and see if the scale of seconds in the third row is just as much, then his calculation is correct. | ||
| − | |style="text-align:right;"|יחל מהשניים ויגרע מאזני השניים השפלים ממאזני<ref group=IV>ממאזני: V397 ומאזני</ref> | + | |style="text-align:right;"|יחל מהשניים ויגרע מאזני השניים השפלים ממאזני השניים העליונים וישמור הנשאר ויראה אם היה כמוהו מאזני השניים בטור השלישי חשבונו אמת‫<ref group=IV>ממאזני: V397 ומאזני</ref> |
|- | |- | ||
|If he sees that he has taken one minute from the minutes and added to the seconds, he adds 6 to the scale of the upper seconds and then subtracts according to the instructions. | |If he sees that he has taken one minute from the minutes and added to the seconds, he adds 6 to the scale of the upper seconds and then subtracts according to the instructions. | ||
| − | |style="text-align:right;"|ואם ראה שלקח ראשון אחד מן הראשונים ושם עם השניים יוסיף על מאזני השניים העליונים ו' ואחר כך יגרע כמשפט‫<ref group=IV>יגרע כמשפט: V397 om.</ref> | + | |style="text-align:right;"|ואם ראה שלקח ראשון אחד מן הראשונים ושם עם השניים <div id="he adds six to the scale of the upper seconds"></div>יוסיף על מאזני השניים העליונים ו‫'‫<ref group=note>[[פירוש_אנונימי_לספר_המספר#He_adds_six_to_the_scale_of_the_upper_seconds|<span style=color:blue>commentary: he adds six to the scale of the upper seconds</span>]]</ref> ואחר כך יגרע כמשפט‫<ref group=IV>יגרע כמשפט: V397 om.</ref> |
|- | |- | ||
|He does with the scales of the minutes as he has done with the seconds. | |He does with the scales of the minutes as he has done with the seconds. | ||
| − | |style="text-align:right;"|ויעשה<ref group=IV>ויעשה: V397 יעשה</ref> | + | |style="text-align:right;"|ויעשה למאזני הראשונים כאשר עשה לשניים‫<ref group=IV>ויעשה: V397 יעשה</ref> |
|- | |- | ||
|If he had to take a degree and convert it to 60 minutes to add them to those that are written there, add 6 to the scale of the minutes that are there, then subtract and proceed according to the instructions. | |If he had to take a degree and convert it to 60 minutes to add them to those that are written there, add 6 to the scale of the minutes that are there, then subtract and proceed according to the instructions. | ||
| − | |style="text-align:right;"|ואם הוצרך לקחת מעלה והשיבה<ref group=IV>והשיבה: V397 והשיב אותו< | + | |style="text-align:right;"|ואם הוצרך לקחת מעלה והשיבה לס' ראשונים לחברם עם הכתובים שם הוסף על מאזני הראשונים שהיו שם ו' ואחר כך תגרע ותעשה כמשפט‫<ref group=IV>והשיבה: V397 והשיב אותו<br> |
| + | לחברם: V397 לחבר אותו</ref> | ||
|- | |- | ||
|Do with the scales of the degrees the same as with the minutes and seconds. | |Do with the scales of the degrees the same as with the minutes and seconds. | ||
| Line 2,666: | Line 4,421: | ||
|- | |- | ||
|If you have taken from the signs one that you have set with the degrees, add 3 to the scale of the degrees that are written, then subtract and proceed according to the instructions. | |If you have taken from the signs one that you have set with the degrees, add 3 to the scale of the degrees that are written, then subtract and proceed according to the instructions. | ||
| − | |style="text-align:right;"|ואם לקחת מן המזלות | + | |style="text-align:right;"|ואם לקחת מן המזלות אחד ששמת אותו עם המעלות הוסף על מאזני המעלות הכתובים בראשונה ג' ואחר כן תגרע ותעשה כמשפט‫<ref group=IV>מן המזלות: V397 מהמזלות<br> |
| + | הוסף על מאזני המעלות: V397 marg.</ref> | ||
|- | |- | ||
|Do with the scales of signs the same as you did with all of them. | |Do with the scales of signs the same as you did with all of them. | ||
| − | |style="text-align:right;"|ועשה במאזני המזלות<ref group=IV>המזלות: V397 המעלות</ref> | + | |style="text-align:right;"|ועשה במאזני המזלות כמשפט שעשית בכל אלה‫<ref group=IV>המזלות: V397 המעלות</ref> |
|- | |- | ||
|If you added 12 to the original signs, add 3 to the scale of the signs that were first written, then subtract and proceed according to the instructions. | |If you added 12 to the original signs, add 3 to the scale of the signs that were first written, then subtract and proceed according to the instructions. | ||
| Line 2,675: | Line 4,431: | ||
|- | |- | ||
|I shall tell you a rule, one thing that is necessary when subtracting: | |I shall tell you a rule, one thing that is necessary when subtracting: | ||
| − | |style="text-align:right;"|כלל אומר לך<ref group=IV>כלל אומר לך: V397 om.< | + | |style="text-align:right;"|כלל אומר לך דבר שהוא צורך למגרעת‫<ref group=IV>כלל אומר לך: V397 om.<br> |
| + | צורך: V397 צריך</ref> | ||
|- | |- | ||
|The last [digit] at the end of the upper row must always be greater than the last [digit] in the lower row, when the rows are the same, the upper [has] the same [number of ranks] as the lower one. | |The last [digit] at the end of the upper row must always be greater than the last [digit] in the lower row, when the rows are the same, the upper [has] the same [number of ranks] as the lower one. | ||
| − | |style="text-align:right;"|לעולם האחרון<ref group=IV>האחרון: V397 אותו< | + | |style="text-align:right;"|לעולם האחרון שבסוף הטור העליון יהיה גדול מהאחרון שבטור השפל כשהטורים שוים שהעליון כמו התחתון‫<ref group=IV>האחרון: V397 אותו<br> |
| + | שבסוף: V397 בסוף<br> | ||
| + | מהאחרון: V397 מאותו האחרון<br> | ||
| + | שבטור: V397 בטור</ref> | ||
|- | |- | ||
|If the rows are not the same, as the upper one is greater than the lower one in respect of the number of digits, as here [igure is missing], return one back and that is enough. | |If the rows are not the same, as the upper one is greater than the lower one in respect of the number of digits, as here [igure is missing], return one back and that is enough. | ||
| − | |style="text-align:right;"|ואם אין הטורים שוים שהעליון<ref group=IV>שהעליון: V397 העליון< | + | |style="text-align:right;"|ואם אין הטורים שוים שהעליון גדול מן התחתון במספר אותיות כזה ישיב האחד אחורנית ודי לו‫<ref group=IV>שהעליון: V397 העליון<br> |
| + | מן התחתון: V397 מ<sup>ה</sup>תחתון<br> | ||
| + | אותיות: V397 אותיות סימנים<br> | ||
| + | ודי לו: V397 ודי לו לכתוב בחלוק והוא צרך לעולם יש לנו לחלק חשבון העליון על התחתון עד שיצא לסוף החשבון יצא לחשבון אם גלגל עליו עוד לא יתחלק דמיון<br> | ||
| + | ב ג ד ה ו ז<br> | ||
| + | ‫0 ב ט ח</ref> | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|דרך אחרת {{#annot:term|181|lNwV}}לגרוע חשבון מחשבון{{#annotend:lNwV}} בהפך שכתוב למעלה שיחל לגרוע מן האחדים‫<ref group=IV>V397 om.</ref> |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<big>דמיון</big> רצינו לגרוע ט' מב' והנו כזו הצורה |
|- | |- | ||
| | | | ||
| Line 2,707: | Line 4,472: | ||
!style="text-align:right;"|<big>השער הה' הוא שער השברים</big> | !style="text-align:right;"|<big>השער הה' הוא שער השברים</big> | ||
|- | |- | ||
| − | |It is known that the one is as a point in a circle; therefore one cannot be a fraction | + | |It is known that the one is as a point in a circle; therefore one cannot be a fraction. |
| − | |style="text-align:right;"|ידוע כי האחד כמו נקודה בתוך {{#annot:term|304|Twc4}}עגולה{{#annotend:Twc4}} על כן לא יתכן להיות האחד נשבר רק בעבור שיקרא הכלל בשם אחד כמו הצורה שהיא כוללת כל הגוף והגוף מורכב משטחים ובעבור זה יעשה האדם מן האחד שברים במחשבה ושברי שברים | + | |style="text-align:right;"|<div id="one_is_as_a_point_in_a_circle">ידוע כי האחד כמו נקודה בתוך {{#annot:term|304|Twc4}}עגולה{{#annotend:Twc4}}</div>‫<ref>[[פירוש_אנונימי_לספר_המספר#One_is_as_a_point_in_a_circle|<span style=color:blue>anonymous commentary: one is as a point in a circle</span>]]; [[Excerpts_Attributed_to_Immanuel_Bonfils#One_is_like_a_point|<span style=color:blue>Bonfils commentary: one is like a point</span>]]</ref> על כן לא יתכן להיות האחד נשבר |
| + | |- | ||
| + | |The whole is named with one name, as the shape represents the entire body. | ||
| + | |style="text-align:right;"|<div id="the_whole_has_one_name">רק בעבור שיקרא הכלל בשם אחד כמו הצורה שהיא כוללת כל הגוף</div> | ||
| + | |- | ||
| + | |The body is composed of surfaces. | ||
| + | |style="text-align:right;"|<div id="the_body_consists_of_surfaces">והגוף מורכב משטחים</div>‫<ref>[[Excerpts_Attributed_to_Immanuel_Bonfils#Consists_of_surfaces|<span style=color:blue>Bonfils commentary: the body consists of surfaces</span>]]</ref> | ||
| + | |- | ||
| + | |Therefore man considers the one in thought as fractions and fraction of fractions . | ||
| + | |style="text-align:right;"|ובעבור זה יעשה האדם מן האחד שברים במחשבה ושברי שברים | ||
| + | |- | ||
| + | |The arithmeticians take all their fractions from a great number so that its fractions are whole units. | ||
| + | |style="text-align:right;"|וחכמי החשבון יקחו כל שבריהם מחשבון גדול שיהיו שבריו אחדים שלמים | ||
|- | |- | ||
| − | | | + | |Thus, they take the half from two. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<div id="arithmeticians_take_half_from_two">על כן יוציאו החצי משנים</div> |
|- | |- | ||
| − | |The analogous number from which they derive is called the "denominator"; | + | |The third from three. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והשליש משלשה |
| + | |- | ||
| + | |And so on to the end of the first rank of the number. | ||
| + | |style="text-align:right;"|וככה עד סוף המערכת הראשונה בחשבון | ||
| + | |- | ||
| + | |The analogous number from which they derive is called the "denominator". | ||
| + | |style="text-align:right;"|<div id="the_analogous_is_called_denominator">והדמיון שיקחו ממנו יקראוהו המורה</div> | ||
| + | |- | ||
| + | |For the product is divided by its square. | ||
| + | |style="text-align:right;"|<div id="the_product_is_divided_by_its_square">כי על מרובעו יחלקו העולה בחשבון</div> | ||
| + | |- | ||
| + | |The remainder that cannot be divided is one part of it or a number of parts that can be named by the name of the units, such as one-quarter, one-third and their similar. | ||
| + | |style="text-align:right;"|<div id="the_remainder_that_cannot_be_divided">והנשאר שלא יתחלק</div> יהיה חלק ממנו או חלקים שיוכל להזכירו בשם האחדים כמו רביעית שלישית והדומה להם | ||
|- | |- | ||
|Sometimes the denominator is a number that has no parts that man can express, because it is a prime number that is not composed, such as 11 or 13, and their like. | |Sometimes the denominator is a number that has no parts that man can express, because it is a prime number that is not composed, such as 11 or 13, and their like. | ||
| Line 2,723: | Line 4,512: | ||
|- | |- | ||
|The one, on the one hand, is not a number, and on the other hand, it is a number. | |The one, on the one hand, is not a number, and on the other hand, it is a number. | ||
| − | |style="text-align:right;"|והנה האחד מפאה אחד איננו מספר ומפאה אחרת הוא מספר | + | |style="text-align:right;"|<div id="one_is_not_a_number">והנה האחד מפאה אחד איננו מספר</div> ומפאה אחרת הוא מספר |
| + | |- | ||
| + | |It is similar to an odd number: | ||
| + | |style="text-align:right;"|<div id="similar_to_an_odd_number">והוא דומה לנפרד</div>‫<ref>[[Excerpts_Attributed_to_Immanuel_Bonfils#It_is_similar_to_an_odd_number|<span style=color:blue>Bonfils commentary: it is similar to an odd number</span>]]</ref> | ||
|- | |- | ||
| − | | | + | |Because when you sum all the odd numbers together successively the squares are generated. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<div id="the_sum_of_odd_numbers">כי בחברך כל הנפרדים</div> זה על זה על הסדר יולדו המרובעים |
|- | |- | ||
|As well as many other matters, which there is no need to mention. | |As well as many other matters, which there is no need to mention. | ||
| Line 2,743: | Line 4,535: | ||
|- | |- | ||
|When there is a need for two fractions that are not of one kind, which are not similar to each other, one looks for each of the fractions: from which number each of them is derived and multiplies one number by the other; the product is the denominator. | |When there is a need for two fractions that are not of one kind, which are not similar to each other, one looks for each of the fractions: from which number each of them is derived and multiplies one number by the other; the product is the denominator. | ||
| − | |style="text-align:right;"|וכאשר יצטרכו לשנים שברים שאינם ממין אחד שלם שלא ידמה זה לזה יבקשו כל אחד מהשברים מאיזה חשבון יצא כל אחד מהם ויכפלו חשבון האחד על האחר והעולה בחבור הוא המורה | + | |style="text-align:right;"|<div id="2_fractions_of_different_kinds">וכאשר יצטרכו לשנים שברים שאינם ממין אחד</div> שלם שלא ידמה זה לזה יבקשו כל אחד מהשברים מאיזה חשבון יצא כל אחד מהם ויכפלו חשבון האחד על האחר והעולה בחבור הוא המורה |
|- | |- | ||
|If there are 3 types, one multiplies the number from which the [first] fraction is derived by the second number from which the second fraction is derived and multiply the product by the number from which the third fraction is derived. | |If there are 3 types, one multiplies the number from which the [first] fraction is derived by the second number from which the second fraction is derived and multiply the product by the number from which the third fraction is derived. | ||
| − | |style="text-align:right;"|ואם היה ג' מינים יכפול החשבון שיצאו ממנו השברים על החשבון השני שיצאו ממנו שברי השני והמחובר יכפלהו על המספר שיצאו ממנו שברי השלישי | + | |style="text-align:right;"|<div id="3_types_of_fractions">ואם היה ג' מינים</div> יכפול החשבון שיצאו ממנו השברים על החשבון השני שיצאו ממנו שברי השני והמחובר יכפלהו על המספר שיצאו ממנו שברי השלישי |
|- | |- | ||
|The same is done if there are 4 types or more: one looks for a common denominator for all. | |The same is done if there are 4 types or more: one looks for a common denominator for all. | ||
| Line 2,764: | Line 4,556: | ||
|- | |- | ||
|First, I shall tell you a rule: that the multiplication of fractions is opposite to the multiplication of integers. | |First, I shall tell you a rule: that the multiplication of fractions is opposite to the multiplication of integers. | ||
| − | |style="text-align:right;"|ואומר לך כלל בתחלה כי כפלי השברים הפך כפלי השלמים | + | |style="text-align:right;"|ואומר לך כלל בתחלה כי <div id="multiplication_of_fractions_is_opposite_to_multiplication_of_integers">כפלי השברים הפך כפלי השלמים</div> |
|- | |- | ||
|Because when it is said: multiply one-half by one-half it is as if it is said: take one-half of one-half; the result is one-quarter. | |Because when it is said: multiply one-half by one-half it is as if it is said: take one-half of one-half; the result is one-quarter. | ||
| Line 2,780: | Line 4,572: | ||
|- | |- | ||
| | | | ||
| − | :*The multiplication of one-quarter by one-quarter is 16 and the | + | :*The multiplication of one-quarter by one-quarter is 16 and the product is one, which is one-half of one-eighth. |
| − | |style="text-align:right;"|וכפל רביעית על רביעית יהיה העולה י"ו והנכפל אחד והנה הוא חצי שמינית | + | |style="text-align:right;"|וכפל רביעית על רביעית יהיה העולה י"ו והנכפל אחד <div id="half_of_an_eighth">והנה הוא חצי שמינית</div>‫<ref>[[Excerpts_Attributed_to_Immanuel_Bonfils#It_is_a_half_of_an_eighth|<span style=color:blue>Bonfils commentary: it is a half of an eighth</span>]]</ref> |
|- | |- | ||
|In this way up to ten and beyond. | |In this way up to ten and beyond. | ||
| Line 2,800: | Line 4,592: | ||
| | | | ||
:*<span style=color:green>common denominator:</span> The denominator is 4. | :*<span style=color:green>common denominator:</span> The denominator is 4. | ||
| − | |style="text-align:right;"|והנה המורה ד' | + | |style="text-align:right;"|והנה המורה ד‫' |
|- | |- | ||
| | | | ||
:*We take 3 for each of the 3 quarters, so the product is 9 <math>\scriptstyle{\color{blue}{\left(\frac{3}{4}\sdot4\right)\times\left(\frac{3}{4}\sdot4\right)=3\times3=9}}</math> | :*We take 3 for each of the 3 quarters, so the product is 9 <math>\scriptstyle{\color{blue}{\left(\frac{3}{4}\sdot4\right)\times\left(\frac{3}{4}\sdot4\right)=3\times3=9}}</math> | ||
| − | |style="text-align:right;"|לקחנו לכל אחד מג' רביעיות ג' והנה הנכפל ט' | + | |style="text-align:right;"|לקחנו לכל אחד מג' רביעיות ג' והנה הנכפל ט‫' |
|- | |- | ||
| | | | ||
| Line 2,822: | Line 4,614: | ||
| | | | ||
:*<span style=color:green>common denominator:</span> The denominator is 5. | :*<span style=color:green>common denominator:</span> The denominator is 5. | ||
| − | |style="text-align:right;"|והנה המורה ה' | + | |style="text-align:right;"|והנה המורה ה‫' |
|- | |- | ||
| | | | ||
| Line 2,865: | Line 4,657: | ||
| | | | ||
::Example. We have taken the one denominator 3, because it was said thirds, and the other denominator 4, because it was said quarters. We multiply the one denominator, which is 3, by the other denominator, which is 4, the result is 12 and it is the required, because we take the ratio of the product to it. We take two for the 2 thirds, because we have taken it from the 3, and three for the 3 quarter, because we have taken it from the 4. We multiply 2 by 3, the result is 6, which is half the product of the denominators. | ::Example. We have taken the one denominator 3, because it was said thirds, and the other denominator 4, because it was said quarters. We multiply the one denominator, which is 3, by the other denominator, which is 4, the result is 12 and it is the required, because we take the ratio of the product to it. We take two for the 2 thirds, because we have taken it from the 3, and three for the 3 quarter, because we have taken it from the 4. We multiply 2 by 3, the result is 6, which is half the product of the denominators. | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<big>דמיון</big> לקחנו המורה האחד ג' בעבור כי אמר שלישיות והמורה האחר ד' בעבור כי אמר רביעיות נכפול המורה האחד שהוא ג' על המורה האחר שהוא ד' ועלו י"ב והוא המבוקש כי העולה נקח ערכו אליו ונקח בעבור הב' שלישיות שנים כי מג' לקחנוהו ומג' רביעיות שלשה כי מד' לקחנוהו ונכפול ב' על ג' עלו ו' והוא חצי מספר הנכפל מהמורים |
|- | |- | ||
| | | | ||
| Line 2,874: | Line 4,666: | ||
| | | | ||
:*<span style=color:green>common denominator:</span> We seek for a common denominator: it is 63 by the multiplication of 7 by 9 <math>\scriptstyle{\color{blue}{7\times9=63}}</math> | :*<span style=color:green>common denominator:</span> We seek for a common denominator: it is 63 by the multiplication of 7 by 9 <math>\scriptstyle{\color{blue}{7\times9=63}}</math> | ||
| − | |style="text-align:right;"|נבקש מורה אחד לשניהם והנו ס"ג בכפל ז' על ט' | + | |style="text-align:right;"|נבקש מורה אחד לשניהם והנו ס"ג בכפל ז' על ט‫' |
|- | |- | ||
| | | | ||
| Line 2,922: | Line 4,714: | ||
:We multiply 84 by 105 and the product by 72. The result of their division is a part of the square of 126; the quotient is 40. | :We multiply 84 by 105 and the product by 72. The result of their division is a part of the square of 126; the quotient is 40. | ||
:<math>\scriptstyle{\color{blue}{\frac{2}{3}\times\frac{5}{6}\times\frac{4}{7}=\frac{84\times105\times72}{126^2}=40}}</math> | :<math>\scriptstyle{\color{blue}{\frac{2}{3}\times\frac{5}{6}\times\frac{4}{7}=\frac{84\times105\times72}{126^2}=40}}</math> | ||
| − | |style="text-align:right;"|כפלנו פ"ד על ק"ה והמחובר על ע"ב והעולה מהם בחלוק הוא חלק ממרובע קכ"ו והחלוק יהיה מ' | + | |style="text-align:right;"|כפלנו פ"ד על ק"ה והמחובר על ע"ב והעולה מהם בחלוק הוא חלק ממרובע קכ"ו והחלוק יהיה מ‫' |
|- | |- | ||
|If we take 3 denominators for them, since they are three types, you do not need the square of the denominator, but take the denominator and divide the product of the numbers by it. | |If we take 3 denominators for them, since they are three types, you do not need the square of the denominator, but take the denominator and divide the product of the numbers by it. | ||
| Line 2,952: | Line 4,744: | ||
*{{#annot:4×⅗|17|uLMJ}}Example: we wish to multiply 4 integers by 3 fifths. | *{{#annot:4×⅗|17|uLMJ}}Example: we wish to multiply 4 integers by 3 fifths. | ||
:<math>\scriptstyle4\times\frac{3}{5}</math> | :<math>\scriptstyle4\times\frac{3}{5}</math> | ||
| − | |style="text-align:right;"|<big>דמיון</big> רצינו לכפול ד' שלמים על ג' חמישיות אחד{{#annotend:uLMJ}} | + | |style="text-align:right;"|<div id="4_by_3_fifths"><big>דמיון</big> רצינו לכפול ד' שלמים על ג' חמישיות אחד</div>{{#annotend:uLMJ}} |
|- | |- | ||
| | | | ||
:*<span style=color:green>common denominator:</span> The denominator is 5. | :*<span style=color:green>common denominator:</span> The denominator is 5. | ||
| − | |style="text-align:right;"|והמורה ה' | + | |style="text-align:right;"|והמורה ה‫' |
|- | |- | ||
| | | | ||
| Line 2,983: | Line 4,775: | ||
| | | | ||
:*First, we multiply the integers by the integers; the result is 20. | :*First, we multiply the integers by the integers; the result is 20. | ||
| − | |style="text-align:right;"|נכפול תחלה השלמים על השלמים עלו כ' | + | |style="text-align:right;"|נכפול תחלה השלמים על השלמים עלו כ‫' |
|- | |- | ||
| | | | ||
| Line 3,028: | Line 4,820: | ||
| | | | ||
:*First, we multiply integers by integers; they are 6. | :*First, we multiply integers by integers; they are 6. | ||
| − | |style="text-align:right;"|נכפול תחלה שלמים על שלמים והם ו' | + | |style="text-align:right;"|נכפול תחלה שלמים על שלמים והם ו‫' |
|- | |- | ||
| | | | ||
| Line 3,036: | Line 4,828: | ||
| | | | ||
::*3 by 3 are 9 | ::*3 by 3 are 9 | ||
| − | |style="text-align:right;"|ג' על ג' והם ט' | + | |style="text-align:right;"|ג' על ג' והם ט‫' |
|- | |- | ||
| | | | ||
::*2 by 4 are 8 | ::*2 by 4 are 8 | ||
| − | |style="text-align:right;"|וב' על ד' והם ח' | + | |style="text-align:right;"|וב' על ד' והם ח‫' |
|- | |- | ||
| | | | ||
| Line 3,085: | Line 4,877: | ||
| | | | ||
:*We also multiply these 3 by the fractions of the number that are 7; the result is 21. We divide it by 8, because there are eighths; the result is 2 integers. So, 20 integers, and 5 eighths remain. We know that the denominator is 40, because you multiply 5 by 8, and these 5 eighths are 25 parts, for you multiply 5 by fifths, which are 5. | :*We also multiply these 3 by the fractions of the number that are 7; the result is 21. We divide it by 8, because there are eighths; the result is 2 integers. So, 20 integers, and 5 eighths remain. We know that the denominator is 40, because you multiply 5 by 8, and these 5 eighths are 25 parts, for you multiply 5 by fifths, which are 5. | ||
| − | |style="text-align:right;"|ונכפול עוד אלו ג' על שברי החשבון שהם ז' יעלו כ"א נחלקם על ח' כי שמיניות הם יעלו ב' שלמים והנה ב' שלמים ונשארו ה' שמיניות ידענו כי המורה הוא מ' כי תכפול ה' על ח' ואלה הה' שמיניות כ"ה חלקים כי תכפול ה' על חמישיות שהם ה' | + | |style="text-align:right;"|ונכפול עוד אלו ג' על שברי החשבון שהם ז' יעלו כ"א נחלקם על ח' כי שמיניות הם יעלו ב' שלמים והנה ב' שלמים ונשארו ה' שמיניות ידענו כי המורה הוא מ' כי תכפול ה' על ח' ואלה הה' שמיניות כ"ה חלקים כי תכפול ה' על חמישיות שהם ה‫' |
|- | |- | ||
| | | | ||
| Line 3,139: | Line 4,931: | ||
| | | | ||
:We now have to beware that the fractions are reversed, because each of the 7 become 11 and each of the 11 become 7. | :We now have to beware that the fractions are reversed, because each of the 7 become 11 and each of the 11 become 7. | ||
| − | |style="text-align:right;"|והנה יש לנו להשמר כי השברים יהיו להפך כי כל אחד מהז' יהיו י"א וכל אחד מהי"א יהיו ז' | + | |style="text-align:right;"|והנה יש לנו להשמר כי השברים יהיו להפך כי כל אחד מהז' יהיו י"א וכל אחד מהי"א יהיו ז‫' |
|- | |- | ||
| | | | ||
| Line 3,209: | Line 5,001: | ||
| | | | ||
:The fractions are numerous; but I shall teach you a short method how to proceed: | :The fractions are numerous; but I shall teach you a short method how to proceed: | ||
| − | |style="text-align:right;"|והנה השברים רבים אך אלמד לך דרך קצרה איך תעשה | + | |style="text-align:right;"|והנה השברים רבים <div id="short_method">אך אלמד לך דרך קצרה איך תעשה</div>‫<ref>[[Excerpts_Attributed_to_Immanuel_Bonfils#But_I_shall_teach_you_a_short_method|<span style=color:blue>Bonfils commentary: but I shall teach you a short method</span>]]</ref> |
|- | |- | ||
| | | | ||
| Line 3,217: | Line 5,009: | ||
| | | | ||
::We multiply 5 by 6; they are 30; also 30 by 7; they are 210; also 210 by 8; the result is one thousand 6 hundred and 80. | ::We multiply 5 by 6; they are 30; also 30 by 7; they are 210; also 210 by 8; the result is one thousand 6 hundred and 80. | ||
| − | |style="text-align:right;"|נכפול ה' על ו' יעלו ל' גם ל' על ז' יהיו ר"י גם ר"י על ח' יעלו אלף ו' מאות ופ' | + | |style="text-align:right;"|נכפול ה' על ו' יעלו ל' גם ל' על ז' יהיו ר"י גם ר"י על ח' יעלו אלף ו' מאות ופ‫' |
|- | |- | ||
| | | | ||
| Line 3,229: | Line 5,021: | ||
| | | | ||
:We multiply one by the other; the number is 10 thousand and 80. We divide this by one thousand 6 hundred and 80; the result is 6 and these 6 are one-fifth of one-eighth of one-seventh, for the seventh is 240, the eighth is 30 and the fifth is 6. | :We multiply one by the other; the number is 10 thousand and 80. We divide this by one thousand 6 hundred and 80; the result is 6 and these 6 are one-fifth of one-eighth of one-seventh, for the seventh is 240, the eighth is 30 and the fifth is 6. | ||
| − | |style="text-align:right;"|נכפול זה על זה יהיה המספר י' (ו') אלפים ופ' (תש"כ) חלקנו זה על אלף ו' מאות ופ' עלו ו' (ד') ואלה הו' (ד') הם חמישית שמינית (חצי ששית) השביעית שהשביעית ר"מ והשמינית ל' והחמישית ו' | + | |style="text-align:right;"|נכפול זה על זה יהיה המספר י' (ו') אלפים ופ' (תש"כ) חלקנו זה על אלף ו' מאות ופ' עלו ו' (ד') ואלה הו' (ד') הם חמישית שמינית (חצי ששית) השביעית שהשביעית ר"מ והשמינית ל' והחמישית ו‫' |
|- | |- | ||
|Now we talk again about the division of the fractions. Do as I have shown you, by turning the fractions into whole units. | |Now we talk again about the division of the fractions. Do as I have shown you, by turning the fractions into whole units. | ||
| − | |style="text-align:right;"|ועתה נשוב לדבר על חלוק השברים עשה כדרך שהראיתיך שתשיב הנשברים אחדים שלמי' | + | |style="text-align:right;"|ועתה נשוב לדבר על חלוק השברים עשה כדרך שהראיתיך שתשיב הנשברים אחדים שלמי‫' |
|- | |- | ||
|If there are integers with the fractions, proceed according to the instructions. | |If there are integers with the fractions, proceed according to the instructions. | ||
| Line 3,314: | Line 5,106: | ||
| | | | ||
:*<span style=color:green>common denominator:</span> We set the denominator as 90. | :*<span style=color:green>common denominator:</span> We set the denominator as 90. | ||
| − | |style="text-align:right;"|נשים המורה צ' | + | |style="text-align:right;"|נשים המורה צ‫' |
|- | |- | ||
| | | | ||
| Line 3,379: | Line 5,171: | ||
| | | | ||
:*The first: the ninth is smaller than the other fractions; we consider them as 3 ninths. The difference between the fifth and ninth is 4 and the difference between the fifth and the seventh is 2. We now multiply 2 by 4; the product is 8. We convert the 7 into one-ninth; they are 4 ninths and one remains. The difference between the seventh and the ninth is 2. So, the amount is 4 ninths and 3 parts that are 3 fifths of one-seventh of one-ninth. | :*The first: the ninth is smaller than the other fractions; we consider them as 3 ninths. The difference between the fifth and ninth is 4 and the difference between the fifth and the seventh is 2. We now multiply 2 by 4; the product is 8. We convert the 7 into one-ninth; they are 4 ninths and one remains. The difference between the seventh and the ninth is 2. So, the amount is 4 ninths and 3 parts that are 3 fifths of one-seventh of one-ninth. | ||
| − | |style="text-align:right;"|'''האחד''' שהתשיעית פחותה משאר השברים האחרים הנה נחשוב כי הם ג' תשיעיות והיתרון שיש בין החמישית והתשיעית ד' והיתרון שיש בין החמישית והשביעית ב' נכפול ב' על ד' יעלו ח' נעשה מן הז' תשיעית אחת יהיו ד' תשיעיות שלמות ונשאר אחד והיתרון בין השביעית (?) והתשיעית (?) ב' והנה הממון הוא ד' תשיעיות וג' חלקים שהם ג' חמישיות שביעית תשיעית | + | |style="text-align:right;"|'''האחד''' שהתשיעית פחותה משאר השברים האחרים הנה נחשוב כי הם ג' תשיעיות <div id="difference_between_fifth_and_ninth">והיתרון שיש בין החמישית והתשיעית ד‫'</div>‫<ref>[[Excerpts_Attributed_to_Immanuel_Bonfils#The_difference_between_the_fifth_and_the_ninth_is_four|<span style=color:blue>Bonfils commentary: the difference between fifth and ninth</span>]]</ref> והיתרון שיש בין החמישית והשביעית ב' נכפול ב' על ד' יעלו ח' נעשה מן הז' תשיעית אחת יהיו ד' תשיעיות שלמות ונשאר אחד והיתרון בין השביעית (?) והתשיעית (?) ב' והנה הממון הוא ד' תשיעיות וג' חלקים שהם ג' חמישיות שביעית תשיעית |
|- | |- | ||
| | | | ||
| Line 3,446: | Line 5,238: | ||
::*We still have to take one-fifth of 3 integers, so we take for each 11 and add to them the additional 2 parts; they are 35 and their fifth is 7. | ::*We still have to take one-fifth of 3 integers, so we take for each 11 and add to them the additional 2 parts; they are 35 and their fifth is 7. | ||
:::<math>\scriptstyle{\color{blue}{\frac{1}{5}\sdot\left(3+\frac{2}{11}\right)=\frac{1}{5}\sdot\frac{\left(3\sdot11\right)+2}{11}=\frac{1}{5}\sdot\frac{35}{11}=\frac{7}{11}}}</math> | :::<math>\scriptstyle{\color{blue}{\frac{1}{5}\sdot\left(3+\frac{2}{11}\right)=\frac{1}{5}\sdot\frac{\left(3\sdot11\right)+2}{11}=\frac{1}{5}\sdot\frac{35}{11}=\frac{7}{11}}}</math> | ||
| − | |style="text-align:right;"|ונשאר לנו לקחת חמישית ג' שלמים והנה נקח לכל אחד י"א ונחבר אליהם הב' חלקים היתרים יהיו ל"ה וחמישיתם ז' | + | |style="text-align:right;"|ונשאר לנו לקחת חמישית ג' שלמים והנה נקח לכל אחד י"א ונחבר אליהם הב' חלקים היתרים יהיו ל"ה וחמישיתם ז‫' |
|- | |- | ||
| | | | ||
| Line 3,477: | Line 5,269: | ||
| | | | ||
:The total is 40. | :The total is 40. | ||
| − | |style="text-align:right;"|והכל מ' | + | |style="text-align:right;"|והכל מ‫' |
|- | |- | ||
|'''<span style=color:green>How Many Problem - Group of People</span>''' | |'''<span style=color:green>How Many Problem - Group of People</span>''' | ||
| Line 3,520: | Line 5,312: | ||
| | | | ||
::<span style=color:green>common denominator:</span> Find the denominator, it is 35, which is the product of 5 by 7. <math>\scriptstyle{\color{blue}{5\sdot7=35}}</math> | ::<span style=color:green>common denominator:</span> Find the denominator, it is 35, which is the product of 5 by 7. <math>\scriptstyle{\color{blue}{5\sdot7=35}}</math> | ||
| − | |style="text-align:right;"|בקש המורה והוא ל"ה שהוא כפל ה' על ז' | + | |style="text-align:right;"|בקש המורה והוא ל"ה שהוא כפל ה' על ז‫' |
|- | |- | ||
| | | | ||
| Line 3,528: | Line 5,320: | ||
| | | | ||
::Its 4 sevenths are 20. <math>\scriptstyle{\color{blue}{x=\frac{4}{7}\sdot35=20}}</math> | ::Its 4 sevenths are 20. <math>\scriptstyle{\color{blue}{x=\frac{4}{7}\sdot35=20}}</math> | ||
| − | |style="text-align:right;"|וד' שביעיותיו כ' | + | |style="text-align:right;"|וד' שביעיותיו כ‫' |
|- | |- | ||
| | | | ||
| Line 3,568: | Line 5,360: | ||
| | | | ||
::Multiply them by 35; they are 70. <math>\scriptstyle{\color{blue}{x=2\sdot35=70}}</math> | ::Multiply them by 35; they are 70. <math>\scriptstyle{\color{blue}{x=2\sdot35=70}}</math> | ||
| − | |style="text-align:right;"|כפלם על ל"ה יהיו ע' | + | |style="text-align:right;"|כפלם על ל"ה יהיו ע‫' |
|- | |- | ||
| | | | ||
| Line 3,601: | Line 5,393: | ||
| | | | ||
:The number of liṭra is 90. | :The number of liṭra is 90. | ||
| − | |style="text-align:right;"|והליט' צ' | + | |style="text-align:right;"|והליט' צ‫' |
|- | |- | ||
|The following reason of subtraction is easy: | |The following reason of subtraction is easy: | ||
| Line 3,751: | Line 5,543: | ||
|- | |- | ||
|So, of sixths remain 33, of the fifths 56, of the fourths 31, of the thirds 24, of the seconds 30, of the minutes 7 and of the degrees also 7. | |So, of sixths remain 33, of the fifths 56, of the fourths 31, of the thirds 24, of the seconds 30, of the minutes 7 and of the degrees also 7. | ||
| − | |style="text-align:right;"|והנה נשארו מן הששיים ג'ג' ומן החמישיים ו'ה' ומהרביעיים א'ג' ומהשלישיים ד'ב' ומהשניים 0ג' ומהראשונים ז' ומהמעלות גם כן ז' | + | |style="text-align:right;"|והנה נשארו מן הששיים ג'ג' ומן החמישיים ו'ה' ומהרביעיים א'ג' ומהשלישיים ד'ב' ומהשניים 0ג' ומהראשונים ז' ומהמעלות גם כן ז‫' |
|- | |- | ||
|The second method is by expression: | |The second method is by expression: | ||
| Line 3,760: | Line 5,552: | ||
|- | |- | ||
|When we do according to the method of the arithmeticians that we convert the upper rows into thirds, the resulting number is: 464643. | |When we do according to the method of the arithmeticians that we convert the upper rows into thirds, the resulting number is: 464643. | ||
| − | |style="text-align:right;"|וכאשר עשינו על דרך חכמי החשבון שהשיבונו הטורים העליונים אל שלישיים עלה החשבון כך ג' ד' ו' ד' ו' ד' | + | |style="text-align:right;"|וכאשר עשינו על דרך חכמי החשבון שהשיבונו הטורים העליונים אל שלישיים עלה החשבון כך ג' ד' ו' ד' ו' ד‫' |
|- | |- | ||
|Likewise, we do it with the lower [rows], the resulting number is: 715451. | |Likewise, we do it with the lower [rows], the resulting number is: 715451. | ||
| − | |style="text-align:right;"|ככה עשינו בשפלים עלה החשבון כך א' ה' ד' ה' א' ז' | + | |style="text-align:right;"|ככה עשינו בשפלים עלה החשבון כך א' ה' ד' ה' א' ז‫' |
|- | |- | ||
|We multiply one by the other, the resulting number is: 332429298993; it has 12 rows. | |We multiply one by the other, the resulting number is: 332429298993; it has 12 rows. | ||
| Line 3,814: | Line 5,606: | ||
| | | | ||
::Such as: 1, 2, 3 | ::Such as: 1, 2, 3 | ||
| − | |style="text-align:right;"|כמו א' ב' ג' | + | |style="text-align:right;"|כמו א' ב' ג‫' |
|- | |- | ||
| | | | ||
| Line 3,822: | Line 5,614: | ||
| | | | ||
::Or: 2, 4, 6 | ::Or: 2, 4, 6 | ||
| − | |style="text-align:right;"|או ב' ד' ו' | + | |style="text-align:right;"|או ב' ד' ו‫' |
|- | |- | ||
| | | | ||
::Or: 3, 6, 9. | ::Or: 3, 6, 9. | ||
| − | |style="text-align:right;"|או ג' ו' ט' | + | |style="text-align:right;"|או ג' ו' ט‫' |
|- | |- | ||
| | | | ||
| Line 3,834: | Line 5,626: | ||
| | | | ||
::Meaning: as the excess of 4 over 2, so is the excess of 6 over 4. | ::Meaning: as the excess of 4 over 2, so is the excess of 6 over 4. | ||
| − | |style="text-align:right;"|פי' כי כיתרון ד' על ב' כן יתרון ו' על ד' | + | |style="text-align:right;"|פי' כי כיתרון ד' על ב' כן יתרון ו' על ד‫' |
|- | |- | ||
| | | | ||
| Line 3,842: | Line 5,634: | ||
| | | | ||
::Such as: 4, 6, 9, | ::Such as: 4, 6, 9, | ||
| − | |style="text-align:right;"|כמו ד' ו' ט' | + | |style="text-align:right;"|כמו ד' ו' ט‫' |
|- | |- | ||
| | | | ||
::Because the ratio of 4 to 6 is as the ratio of 6 to 9. | ::Because the ratio of 4 to 6 is as the ratio of 6 to 9. | ||
| − | |style="text-align:right;"|כי ערך ד' אל ו' כמו ערך ו' אל ט' | + | |style="text-align:right;"|כי ערך ד' אל ו' כמו ערך ו' אל ט‫' |
|- | |- | ||
| | | | ||
| Line 3,865: | Line 5,657: | ||
| | | | ||
:*Example: The ratio of 4 to 6 is as the ratio of 8 to 12. | :*Example: The ratio of 4 to 6 is as the ratio of 8 to 12. | ||
| − | |style="text-align:right;"|דמיון ערך ד' על ו' כערך ח' אל י"ב | + | |style="text-align:right;"|<div id="ratio_of_4_6_8">דמיון ערך ד' על ו' כערך ח' אל י"ב</div>‫<ref group=note>[[Excerpts_Attributed_to_Immanuel_Bonfils#Example:_The_ratio_of_4_to_.5B6.5D|<span style=color:blue>commentary: example: The ratio of 4 to 6</span>]]</ref> |
|- | |- | ||
| | | | ||
| Line 3,885: | Line 5,677: | ||
| | | | ||
:This is a very wonderful science, because its proportions are composed of the arithmetic and the geometric proportions. | :This is a very wonderful science, because its proportions are composed of the arithmetic and the geometric proportions. | ||
| − | |style="text-align:right;"|והיא חכמה מפוארת מאד כי ערכיה מורכבים מערכי החשבון וערכי המדות | + | |style="text-align:right;"|והיא חכמה מפוארת מאד <div id="its_proportions_are_composed">כי ערכיה מורכבים מערכי החשבון וערכי המדות</div>‫<ref group=note>[[Excerpts_Attributed_to_Immanuel_Bonfils#Its_proportions_are_composed|<span style=color:blue>commentary: its proportions are composed</span>]]</ref> |
|- | |- | ||
| | | | ||
:Since, the ratio between the first and the mean to the ratio between mean and last is always as the ratio of the first number to the last number. | :Since, the ratio between the first and the mean to the ratio between mean and last is always as the ratio of the first number to the last number. | ||
| − | |style="text-align:right;"|כי לעולם יהיה הערך שבין הראשון לתיכון אל ערך שיהיה בין התיכון <ref>MS N2561 begin.</ref>לאחרון כערך המספר הראשון למספר האחרון | + | :<math>\scriptstyle{\color{OliveGreen}{\left(a_2-a_1\right):\left(a_3-a_2\right)=a_1:a_3}}</math> |
| + | |style="text-align:right;"|<div id="ratio_between_first_and_last">כי לעולם יהיה הערך שבין הראשון לתיכון אל ערך שיהיה בין התיכון <ref>MS N2561 begin.</ref>לאחרון כערך המספר הראשון למספר האחרון</div>‫<ref group=note>[[Excerpts_Attributed_to_Immanuel_Bonfils#Because_the ratio_is_always|<span style=color:blue>commentary: because the ratio is always</span>]]</ref> | ||
|- | |- | ||
| | | | ||
::Example: 2, 3, 6. | ::Example: 2, 3, 6. | ||
| − | |style="text-align:right;"|דמיון ב' ג' ו' | + | |style="text-align:right;"|דמיון ב' ג' ו‫' |
|- | |- | ||
| | | | ||
::We know, that the ratio between 2 and 3 is 1 and the ratio between 3 and 6 is three; and so is the ratio of 2 to 6. | ::We know, that the ratio between 2 and 3 is 1 and the ratio between 3 and 6 is three; and so is the ratio of 2 to 6. | ||
| − | |style="text-align:right;"|ידענו כי הערך שבין ב' וג' אחד והערך שבין ג' ובין ו' שלשה וככה ערך ב' אל ו' | + | |style="text-align:right;"|ידענו כי הערך שבין ב' וג' אחד והערך שבין ג' ובין ו' שלשה וככה ערך ב' אל ו‫' |
|- | |- | ||
| | | | ||
::Another example: 3, 4, 6; or, if you wish, 20, 30, 60. | ::Another example: 3, 4, 6; or, if you wish, 20, 30, 60. | ||
| − | |style="text-align:right;"|דמיון אחר ג' ד' ו' או אם תרצה כ' ל' ס' | + | |style="text-align:right;"|דמיון אחר ג' ד' ו' או אם תרצה כ' ל' ס‫' |
|- | |- | ||
| | | | ||
::The ratio between 3 and 4 is one and the ratio between 4 and 6 is two; hence it is its double and so is 6 to 3. | ::The ratio between 3 and 4 is one and the ratio between 4 and 6 is two; hence it is its double and so is 6 to 3. | ||
| − | |style="text-align:right;"|והנה הערך שבין ג' וד' אחד והערך שבין ד' וו' שנים והנה הוא כפלו וככה ו' אל ג' | + | ::<math>\scriptstyle{\color{blue}{\left(4-3\right):\left(6-4\right)=3:6}}</math> |
| + | |style="text-align:right;"|והנה הערך שבין ג' וד' אחד והערך שבין ד' וו' שנים והנה הוא כפלו וככה ו' אל ג‫' | ||
|- | |- | ||
|If you know two numbers, you can extract the third. | |If you know two numbers, you can extract the third. | ||
| Line 3,912: | Line 5,706: | ||
| | | | ||
:*For, if you know the first and second and you do not know the third, multiply the first by the second, then divide the product by the first after you have subtracted from it, meaning from the first, the difference between it and the second. | :*For, if you know the first and second and you do not know the third, multiply the first by the second, then divide the product by the first after you have subtracted from it, meaning from the first, the difference between it and the second. | ||
| − | |style="text-align:right;"|כי אם ידעת הראשון והשני ולא תדע השלישי כפול הראשון על השני והעולה חלקנו על הראשון אחר שתגרע ממנו פי' מהראשון היתרון שיש בינו ובין השני | + | |style="text-align:right;"|<div id="the_third_is_unknown">כי אם ידעת הראשון והשני ולא תדע השלישי</div> כפול הראשון על השני והעולה חלקנו על הראשון אחר שתגרע ממנו פי' מהראשון היתרון שיש בינו ובין השני‫<ref group=note>[[Excerpts_Attributed_to_Immanuel_Bonfils#And_you_do_not_know_the_third:_multiply_the_first_by_the_second|<span style=color:blue>commentary: and you do not know the third: multiply the first by the second</span>]]</ref> |
|- | |- | ||
| | | | ||
| Line 3,921: | Line 5,715: | ||
::*In the second example: the product of 3 by 4 is 12 and the difference between 3 and 4 is 1. We subtract it from 3, which is the first; 2 remain. We divide 12 by this; they are 6 for each and it is the third. | ::*In the second example: the product of 3 by 4 is 12 and the difference between 3 and 4 is 1. We subtract it from 3, which is the first; 2 remain. We divide 12 by this; they are 6 for each and it is the third. | ||
|style="text-align:right;"|ובדמיון השני כפל ג' על ד' היו י"ב והיתרון בין ג' וד' אחד<br> | |style="text-align:right;"|ובדמיון השני כפל ג' על ד' היו י"ב והיתרון בין ג' וד' אחד<br> | ||
| − | גרענוהו מג' שהוא הראשון ונשארו ב'<br> | + | גרענוהו מג' שהוא הראשון ונשארו ב‫'<br> |
חלקנו עליו י"ב והם ו' לכל אחד והוא השלישי | חלקנו עליו י"ב והם ו' לכל אחד והוא השלישי | ||
|- | |- | ||
| Line 3,930: | Line 5,724: | ||
| | | | ||
::*In the first example: we multiply 3 by 6; the product is 18. The difference between the second and third is 3, we add it to 6; it is 9. We divide 18 by it; the result is 2 and it is the first. | ::*In the first example: we multiply 3 by 6; the product is 18. The difference between the second and third is 3, we add it to 6; it is 9. We divide 18 by it; the result is 2 and it is the first. | ||
| − | |style="text-align:right;"|והנה בדמיון הראשון כפלנו ג' על ו' והעולה י"ח והיתרון שבין השני והשלישי שלשה חברנום אל ו' והיו ט'<br> | + | |style="text-align:right;"|והנה בדמיון הראשון כפלנו ג' על ו' והעולה י"ח והיתרון שבין השני והשלישי שלשה חברנום אל ו' והיו ט‫'<br> |
חלקנו י"ח עליו עלו ב' והוא הראשון | חלקנו י"ח עליו עלו ב' והוא הראשון | ||
|- | |- | ||
| Line 3,951: | Line 5,745: | ||
::*In the other example: we multiply 3 by 6; the result is 18. We divide by 9, which is the sum of both; the quotient is 2. We double this; the result is 4 and this is the second number that is sought. | ::*In the other example: we multiply 3 by 6; the result is 18. We divide by 9, which is the sum of both; the quotient is 2. We double this; the result is 4 and this is the second number that is sought. | ||
|style="text-align:right;"|ובדמיון האחר כפלנו ג' על ו' עלו י"ח<br> | |style="text-align:right;"|ובדמיון האחר כפלנו ג' על ו' עלו י"ח<br> | ||
| − | חלקנו על ט' שהוא המחובר משניהם עלו ב'<br> | + | חלקנו על ט' שהוא המחובר משניהם עלו ב‫'<br> |
כפלנוהו ועלה ד' והוא המספר השני המבוקש | כפלנוהו ועלה ד' והוא המספר השני המבוקש | ||
|- | |- | ||
| | | | ||
*Likewise also for the geometric proportions: If we do not know one of the four, we can extract it from the 3: | *Likewise also for the geometric proportions: If we do not know one of the four, we can extract it from the 3: | ||
| − | |style="text-align:right;"|וככה בערכי המדות אם לא ידענו האחד מן הד' נוכל להוציאו מן הג' | + | |style="text-align:right;"|וככה בערכי המדות אם לא ידענו האחד מן הד' נוכל להוציאו מן הג‫' |
|- | |- | ||
| | | | ||
| Line 3,972: | Line 5,766: | ||
| | | | ||
|style="text-align:right;"|ועל זה הדרך תעשה בכל השאלות ויש לך להשמר באיזה מקום תשים הגלגל | |style="text-align:right;"|ועל זה הדרך תעשה בכל השאלות ויש לך להשמר באיזה מקום תשים הגלגל | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| Line 4,502: | Line 6,294: | ||
|{{#annot:one worker|612|UtpS}}Question: Reuven hired Shimon to work with him for 17 days and he will pay him 11 pešuṭim, but he worked 9 days. | |{{#annot:one worker|612|UtpS}}Question: Reuven hired Shimon to work with him for 17 days and he will pay him 11 pešuṭim, but he worked 9 days. | ||
:<math>\scriptstyle\frac{17}{11}=\frac{9}{X}</math> | :<math>\scriptstyle\frac{17}{11}=\frac{9}{X}</math> | ||
| − | |style="text-align:right;"|<big>שאלה</big> ראובן שכר שמעון שיעבוד עמו י"ז ימים ויתן לו י"א פשו' והנה עבד ט' ימים{{#annotend:UtpS}} | + | |style="text-align:right;"|<big>שאלה</big> <div id="Reuven_hired_Shimon">ראובן שכר שמעון</div> שיעבוד עמו י"ז ימים ויתן לו י"א פשו' והנה עבד ט' ימים{{#annotend:UtpS}}‫<ref group=note>[[Excerpts_Attributed_to_Immanuel_Bonfils#Question:_Reuven_hired_Shimon|<span style=color:blue>commentary: question: Reuven hired Shimon</span>]]</ref> |
|- | |- | ||
| | | | ||
| Line 5,700: | Line 7,492: | ||
|- | |- | ||
| | | | ||
| − | *<span style=color: | + | *<span style=color:green>'''Definition of a square number:'''</span> {{#annot:definition|86|2NJw}}the square [number] is the product of the multiplication of a root by itself |
|style="text-align:right;"|והנה המרובע הוא המחובר מכפל שורש על עצמו{{#annotend:2NJw}} | |style="text-align:right;"|והנה המרובע הוא המחובר מכפל שורש על עצמו{{#annotend:2NJw}} | ||
|- | |- | ||
| | | | ||
::Such as 9: since it is a quadrilateral whose length is the same as its breadth and its root is 3. | ::Such as 9: since it is a quadrilateral whose length is the same as its breadth and its root is 3. | ||
| − | |style="text-align:right;"|כמו ט' כי הוא מרובע ארכו כרחבו ושרשו ג' | + | |style="text-align:right;"|כמו ט' כי הוא מרובע ארכו כרחבו ושרשו ג‫' |
|- | |- | ||
|There are numbers that have no true [rational] root at all, and they are the majority. | |There are numbers that have no true [rational] root at all, and they are the majority. | ||
| Line 5,712: | Line 7,504: | ||
| | | | ||
::Such as 2 in the first rank, also 3, 5, 6, 7 and 8. | ::Such as 2 in the first rank, also 3, 5, 6, 7 and 8. | ||
| − | |style="text-align:right;"|כמו ב' במעלה הראשונה גם ג' וה' וו' וז' וח' | + | |style="text-align:right;"|כמו ב' במעלה הראשונה גם ג' וה' וו' וז' וח‫' |
|- | |- | ||
|The square of an integer is always greater than the root. | |The square of an integer is always greater than the root. | ||
| Line 5,718: | Line 7,510: | ||
|- | |- | ||
|Vice versa for the squares of fractions. | |Vice versa for the squares of fractions. | ||
| − | |style="text-align:right;"|והפך הדבר במרובעי' הנשברי' | + | |style="text-align:right;"|והפך הדבר במרובעי' הנשברי‫' |
|- | |- | ||
|This is because when multiplying a fraction by a fraction, the product is less than the multiplied fraction. | |This is because when multiplying a fraction by a fraction, the product is less than the multiplied fraction. | ||
| Line 5,724: | Line 7,516: | ||
|- | |- | ||
|The one alone is both root and square; because it is between the fractions and the integers. | |The one alone is both root and square; because it is between the fractions and the integers. | ||
| − | |style="text-align:right;"|והיה האחד לבדו שורש ומרובע כי הוא בין השברי' והשלמי' | + | |style="text-align:right;"|והיה האחד לבדו שורש ומרובע כי הוא בין השברי' והשלמי‫' |
|- | |- | ||
| | | | ||
| Line 5,731: | Line 7,523: | ||
| | | | ||
|- | |- | ||
| − | |First, we shall give test methods: | + | |First, we shall give scales [= test methods]: |
|style="text-align:right;"|והנה נתן בתחלה מאזנים | |style="text-align:right;"|והנה נתן בתחלה מאזנים | ||
|- | |- | ||
|Observe: | |Observe: | ||
| − | *If the | + | *If the scales of the square number is not the same as the scales of the product of the root by itself the number is not a square. |
|style="text-align:right;"|הסתכל אם לא היו מאזני המרובע ככפל מאזני השרש על עצמו אין המספר מרובע | |style="text-align:right;"|הסתכל אם לא היו מאזני המרובע ככפל מאזני השרש על עצמו אין המספר מרובע | ||
|- | |- | ||
| Line 5,747: | Line 7,539: | ||
|- | |- | ||
| | | | ||
| − | ::Its | + | ::Its scales are 9; the root is 12, its scales are 3 and its product by itself is 9. |
| − | |style="text-align:right;"|והמאזנים ט' והשרש י"ב ומאזניו ג' וכפל על עצמו הוא ט' | + | |style="text-align:right;"|והמאזנים ט' והשרש י"ב ומאזניו ג' וכפל על עצמו הוא ט‫' |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|והוא מאזני השרש ת' | + | |style="text-align:right;"|והוא מאזני השרש ת‫' |
|- | |- | ||
| − | | | + | |Other scales: |
|style="text-align:right;"|מאזנים אחרים | |style="text-align:right;"|מאזנים אחרים | ||
|- | |- | ||
| | | | ||
| − | *If the | + | *If the scales of the number are 2, 3, 5, 6 or 8, it is not a square |
|style="text-align:right;"|אם מאזני המספר ב' או ג' או ה' או ו' או ח' איננו מרובע | |style="text-align:right;"|אם מאזני המספר ב' או ג' או ה' או ו' או ח' איננו מרובע | ||
|- | |- | ||
| | | | ||
| − | *But, if the | + | *But, if the scales are one of the square numbers that are in the first rank, which are 1, 4, or 9, as well as 7, it can be a square. |
|style="text-align:right;"|ואם היו המאזנים אחד מהמרובעים שהם במעלה הראשונה שהם א' או ד' או ט' גם ז' עמהם יתכן להיות מרובע | |style="text-align:right;"|ואם היו המאזנים אחד מהמרובעים שהם במעלה הראשונה שהם א' או ד' או ט' גם ז' עמהם יתכן להיות מרובע | ||
|- | |- | ||
| − | | | + | |Other scales: |
|style="text-align:right;"|מאזנים אחרים | |style="text-align:right;"|מאזנים אחרים | ||
|- | |- | ||
| Line 5,775: | Line 7,567: | ||
|style="text-align:right;"|ואם היה אחד מן המרובעים א' או ד' או ט' או מן המתגלגלים שהם ה' ו' יתכן שיהיה המספר מרובע | |style="text-align:right;"|ואם היה אחד מן המרובעים א' או ד' או ט' או מן המתגלגלים שהם ה' ו' יתכן שיהיה המספר מרובע | ||
|- | |- | ||
| − | | | + | |Other scales that are reliable: |
|style="text-align:right;"|מאזנים אחרים שהם מאזני צדק | |style="text-align:right;"|מאזנים אחרים שהם מאזני צדק | ||
|- | |- | ||
| | | | ||
*If you find one in the first rank of the sought number, know that there is one or 9 in the root. | *If you find one in the first rank of the sought number, know that there is one or 9 in the root. | ||
| − | |style="text-align:right;"|אם מצאת במספר המבוקש מן המעלה הראשונה אחד דע שיש בשורש אחד או ט' | + | |style="text-align:right;"|אם מצאת במספר המבוקש מן המעלה הראשונה אחד דע שיש בשורש אחד או ט‫' |
|- | |- | ||
| | | | ||
*If there is a 4 in the [square] number, know that there is a 2 or 8 in the root. | *If there is a 4 in the [square] number, know that there is a 2 or 8 in the root. | ||
| − | |style="text-align:right;"|ואם היה במספר ד' דע שיש בשורש ב' או ח' | + | |style="text-align:right;"|ואם היה במספר ד' דע שיש בשורש ב' או ח‫' |
|- | |- | ||
| | | | ||
*If there is a 6 in the [square] number, know that there is a 4 or 6 in the root. | *If there is a 6 in the [square] number, know that there is a 4 or 6 in the root. | ||
| − | |style="text-align:right;"|ואם היה במספר ו' דע שיש בשרש ד' או ו' | + | |style="text-align:right;"|ואם היה במספר ו' דע שיש בשרש ד' או ו‫' |
|- | |- | ||
| | | | ||
*If there is a 9 in the [square] number, know that there is a 3 or 7 in the root. | *If there is a 9 in the [square] number, know that there is a 3 or 7 in the root. | ||
| − | |style="text-align:right;"|ואם במספר ט' דע כי יש בשרש ג' או ז' | + | |style="text-align:right;"|ואם במספר ט' דע כי יש בשרש ג' או ז‫' |
|- | |- | ||
| | | | ||
*If there is a 5 in the square number, know that there is a 5 in the root. | *If there is a 5 in the square number, know that there is a 5 in the root. | ||
| − | |style="text-align:right;"|ואם במרובע ה' דע שיש בשרש ה' | + | |style="text-align:right;"|ואם במרובע ה' דע שיש בשרש ה‫' |
|- | |- | ||
|Now I shall give you a way by which you can know which of the two mentioned [digits] is in the root. | |Now I shall give you a way by which you can know which of the two mentioned [digits] is in the root. | ||
| Line 5,802: | Line 7,594: | ||
|- | |- | ||
|Now, note that the square numbers that are in the first rank are 3, which are 1, 4 9, | |Now, note that the square numbers that are in the first rank are 3, which are 1, 4 9, | ||
| − | |style="text-align:right;"|ועתה שים לבך כי המרובעים במעלה הראשונה הם ג' והם א' ד' ט' | + | |style="text-align:right;"|ועתה שים לבך כי המרובעים במעלה הראשונה הם ג' והם א' ד' ט‫' |
|- | |- | ||
|In the second rank they are 6, which are 16, 25, 36, 49, 64, 81. | |In the second rank they are 6, which are 16, 25, 36, 49, 64, 81. | ||
| Line 5,844: | Line 7,636: | ||
|- | |- | ||
|Subtract the square from the sought number, after making sure that you take only the preceding square, which is the closest to the number, see the difference between the square [and the sought number] and divide it by double the root of the preceding square. Make it so that you do not give everything you can for it, but leave a little bit of it so that you can still take the square of the quotient of it. When you see that the difference between the preceding square [and the sought number] is as much as the quotient, then you know that the square number is true. | |Subtract the square from the sought number, after making sure that you take only the preceding square, which is the closest to the number, see the difference between the square [and the sought number] and divide it by double the root of the preceding square. Make it so that you do not give everything you can for it, but leave a little bit of it so that you can still take the square of the quotient of it. When you see that the difference between the preceding square [and the sought number] is as much as the quotient, then you know that the square number is true. | ||
| − | *<math>\scriptstyle a^2+b</math>: | + | *<math>\scriptstyle{\color{OliveGreen}{a^2+b}}</math>: |
| − | :<math>\scriptstyle n<\frac{b}{2a}\longrightarrow \left(a+n\right)^2=a^2+2\sdot a\sdot n+n^2</math> | + | :<math>\scriptstyle{\color{OliveGreen}{n<\frac{b}{2a}\longrightarrow \left(a+n\right)^2=a^2+2\sdot a\sdot n+n^2}}</math> |
|style="text-align:right;"|חסר המרובע מהמספר המבוקש אחר שתשמור שלא תקח לעולם כי אם המרבע שעבר שהוא קרוב אל מספר וראה המרחק שבין המרבע וחלקהו על כפל שרש המרבע שעבר וככה עשה שלא תתן לו כל מה שתוכל רק הנח ממנו שתוכל לקחת מרבע מה שעלה בחלוק וכאשר תראה שיהיה המרחק בין המרובע שעבר כמספר מה שעלה בחלוק אז תדע שהמרבע אמת | |style="text-align:right;"|חסר המרובע מהמספר המבוקש אחר שתשמור שלא תקח לעולם כי אם המרבע שעבר שהוא קרוב אל מספר וראה המרחק שבין המרבע וחלקהו על כפל שרש המרבע שעבר וככה עשה שלא תתן לו כל מה שתוכל רק הנח ממנו שתוכל לקחת מרבע מה שעלה בחלוק וכאשר תראה שיהיה המרחק בין המרובע שעבר כמספר מה שעלה בחלוק אז תדע שהמרבע אמת | ||
|- | |- | ||
|If the sought number is less than the analogous square number, see how much the difference between your number and the succeeding square number is. Since you have to give it a little bit more than you can because your number precedes the analogous squares, as I will explain later, do not add the square of the quotient to your number. See if the number is as much as double the root multiplied, then you know that your number is true. | |If the sought number is less than the analogous square number, see how much the difference between your number and the succeeding square number is. Since you have to give it a little bit more than you can because your number precedes the analogous squares, as I will explain later, do not add the square of the quotient to your number. See if the number is as much as double the root multiplied, then you know that your number is true. | ||
| − | *<math>\scriptstyle a^2-b</math>: | + | *<math>\scriptstyle{\color{OliveGreen}{a^2-b}}</math>: |
| − | :<math>\scriptstyle \frac{b}{2a}<n\longrightarrow \left(a-n\right)^2=a^2-2\sdot a\sdot n+n^2</math> | + | :<math>\scriptstyle{\color{OliveGreen}{\frac{b}{2a}<n\longrightarrow \left(a-n\right)^2=a^2-2\sdot a\sdot n+n^2}}</math> |
|style="text-align:right;"|ואם היה המספר המבוקש פחות מהמרבע הנמשל ראה כמה מרחק בין מספרך ובין המרבע הבא<br> | |style="text-align:right;"|ואם היה המספר המבוקש פחות מהמרבע הנמשל ראה כמה מרחק בין מספרך ובין המרבע הבא<br> | ||
לפי שאתה צריך לתן לו מעט יותר ממה שתוכל בעבור היות מספרך לפני המרבע הנמשל כאשר אפרש ואל תכניס במספרך מרובע החלוק<br> | לפי שאתה צריך לתן לו מעט יותר ממה שתוכל בעבור היות מספרך לפני המרבע הנמשל כאשר אפרש ואל תכניס במספרך מרובע החלוק<br> | ||
| Line 5,856: | Line 7,648: | ||
|- | |- | ||
|If it is 1, subtract one from tens, then 9 remain. | |If it is 1, subtract one from tens, then 9 remain. | ||
| − | |style="text-align:right;"|כי אם היה אחד חסר מן העשרות אחד וישארו ט' | + | |style="text-align:right;"|כי אם היה אחד חסר מן העשרות אחד וישארו ט‫' |
|- | |- | ||
|In this way you can know: if you find 1 or 9 in the square, then it must be in the root, as you will see from the examples. | |In this way you can know: if you find 1 or 9 in the square, then it must be in the root, as you will see from the examples. | ||
| Line 5,872: | Line 7,664: | ||
והנה מאה הוא המרבע שעבר ושרשו י'<br> | והנה מאה הוא המרבע שעבר ושרשו י'<br> | ||
כי כן אמרנו מה שהם במעלה הראשונה אחדי' יהיו במעלה השלישית עשרות<br> | כי כן אמרנו מה שהם במעלה הראשונה אחדי' יהיו במעלה השלישית עשרות<br> | ||
| − | נחסר המרבע מחשבוננו ונשארו ק'<br> | + | נחסר המרבע מחשבוננו ונשארו ק‫'<br> |
וכבר אמרנו כי השרש י' וכפלו כ' והנה אם חלקנו ק' על כ' ונתן לו ה' לא ישאר כלום שנוכל לקחת ממנו מרבע מה שעלה בחלוק | וכבר אמרנו כי השרש י' וכפלו כ' והנה אם חלקנו ק' על כ' ונתן לו ה' לא ישאר כלום שנוכל לקחת ממנו מרבע מה שעלה בחלוק | ||
|- | |- | ||
| | | | ||
::Therefore we give it 4. Multiplied by 20 it is 80, so 20 remain. We subtract from it 16, which the square of the quotient; 4 remain. We subtract this from two hundred, 196 remain and this is the square number closest to 200. | ::Therefore we give it 4. Multiplied by 20 it is 80, so 20 remain. We subtract from it 16, which the square of the quotient; 4 remain. We subtract this from two hundred, 196 remain and this is the square number closest to 200. | ||
| − | |style="text-align:right;"|והנה נתן לו ד' כפולים על כ' הם פ' נשארו כ' נחסר ממנו י"ו שהוא מרובע מה שעלה בחלוק ישאר ד'<br> | + | |style="text-align:right;"|והנה נתן לו ד' כפולים על כ' הם פ' נשארו כ' נחסר ממנו י"ו שהוא מרובע מה שעלה בחלוק ישאר ד‫'<br> |
נחסרנו מהמאתים ישארו קצ"ו וזהו המרבע הקרוב אל מאתים | נחסרנו מהמאתים ישארו קצ"ו וזהו המרבע הקרוב אל מאתים | ||
|- | |- | ||
| Line 5,901: | Line 7,693: | ||
| | | | ||
:::The excess of the analogous square number is [96]. The double of the root of the analogous square number is 20; we multiply it by 4, the result is 80; we add to it the square of 4, which is 16, it is 96, hence this method is correct. | :::The excess of the analogous square number is [96]. The double of the root of the analogous square number is 20; we multiply it by 4, the result is 80; we add to it the square of 4, which is 16, it is 96, hence this method is correct. | ||
| − | |style="text-align:right;"|והנה המרחק מהמרבע הנמשל ק' וכפל [שורש] מרובע הנמשל כ'<br> | + | |style="text-align:right;"|והנה המרחק מהמרבע הנמשל ק' וכפל [שורש] מרובע הנמשל כ‫'<br> |
| − | כפלנוהו על ד' עלו פ'<br> | + | כפלנוהו על ד' עלו פ‫'<br> |
נוסיף עליו מרובע ד' שהוא י"ו יהיו צ"ו והוא דרך אמת | נוסיף עליו מרובע ד' שהוא י"ו יהיו צ"ו והוא דרך אמת | ||
|- | |- | ||
| Line 5,911: | Line 7,703: | ||
| | | | ||
::3 hundred is closer to 4 hundred, which is an analogous square number in this rank, than to the first square, which is 100. | ::3 hundred is closer to 4 hundred, which is an analogous square number in this rank, than to the first square, which is 100. | ||
| − | |style="text-align:right;"|וג' מאות יותר קרובי' אל ד' מאות שהוא מרבע הנמשל במעלה הזאת ממרובע הראשון שהוא ק' | + | |style="text-align:right;"|וג' מאות יותר קרובי' אל ד' מאות שהוא מרבע הנמשל במעלה הזאת ממרובע הראשון שהוא ק‫' |
|- | |- | ||
| | | | ||
::We consider the excess of the succeeding square number, which is 100: We know that the root of 4 hundred is 20 and its double is 40. We divide 1OO by it and give it a little more than we can because the number precedes the square. If we give it 2, 20 remain, so we give it 3. | ::We consider the excess of the succeeding square number, which is 100: We know that the root of 4 hundred is 20 and its double is 40. We divide 1OO by it and give it a little more than we can because the number precedes the square. If we give it 2, 20 remain, so we give it 3. | ||
::<math>\scriptstyle{\color{blue}{\frac{20^2-300}{2\sdot20}=\frac{400-300}{40}=\frac{100}{40}=2+\frac{20}{100}<3\longrightarrow n=3}}</math> | ::<math>\scriptstyle{\color{blue}{\frac{20^2-300}{2\sdot20}=\frac{400-300}{40}=\frac{100}{40}=2+\frac{20}{100}<3\longrightarrow n=3}}</math> | ||
| − | |style="text-align:right;"|והנה נסתכל המרחק מהמרבע הבא והוא ק' וידענו כי שרש ד' מאות הוא כ' וכפלו מ'<br> | + | |style="text-align:right;"|והנה נסתכל המרחק מהמרבע הבא והוא ק' וידענו כי שרש ד' מאות הוא כ' וכפלו מ‫'<br> |
נחלוק ק' עליו ונתן לו מעט יותר ממה שנוכל בעבור היות המספר לפני המרובע<br> | נחלוק ק' עליו ונתן לו מעט יותר ממה שנוכל בעבור היות המספר לפני המרובע<br> | ||
| − | והנה אם נתן לו ב' ישאר כ' על כן נתן לו ג' | + | והנה אם נתן לו ב' ישאר כ' על כן נתן לו ג‫' |
|- | |- | ||
| | | | ||
| Line 5,941: | Line 7,733: | ||
|- | |- | ||
|Similarly, you can deduce if you find 4 in the square, whether there is 2 or 8 in the square root. | |Similarly, you can deduce if you find 4 in the square, whether there is 2 or 8 in the square root. | ||
| − | |style="text-align:right;"|ובזה תוכל להפריש אם מצאת במרובע ד' אם יש בשרש ב' או ח' | + | |style="text-align:right;"|ובזה תוכל להפריש אם מצאת במרובע ד' אם יש בשרש ב' או ח‫' |
|- | |- | ||
|likewise, if there is 6 in the square, you can know whether there must be 4 or 6 in the root. | |likewise, if there is 6 in the square, you can know whether there must be 4 or 6 in the root. | ||
| − | |style="text-align:right;"|וככה אם יש במרובע ו' תדע אם ראוי להיות בשרש ד' או ו' | + | |style="text-align:right;"|וככה אם יש במרובע ו' תדע אם ראוי להיות בשרש ד' או ו‫' |
|- | |- | ||
|Now I shall reveal to you the secret why this is so. | |Now I shall reveal to you the secret why this is so. | ||
| Line 5,959: | Line 7,751: | ||
|- | |- | ||
|The square of 2 is 4 and there is 4 in the square of 8. | |The square of 2 is 4 and there is 4 in the square of 8. | ||
| − | |style="text-align:right;"|ומרובע ב' ד' וככה יש במרובע ח' ד' | + | |style="text-align:right;"|ומרובע ב' ד' וככה יש במרובע ח' ד‫' |
|- | |- | ||
|The same applies to 3 and 7; 4 and 6. | |The same applies to 3 and 7; 4 and 6. | ||
| − | |style="text-align:right;"|וכן דרך ג' אל ז' וד' עם ו' | + | |style="text-align:right;"|וכן דרך ג' אל ז' וד' עם ו‫' |
|- | |- | ||
|Thus, the number 5 is left as a mean, therefore it is recurring around itself. | |Thus, the number 5 is left as a mean, therefore it is recurring around itself. | ||
| Line 5,970: | Line 7,762: | ||
::You can extract the mentioned square by the first method I have mentioned for the square number 196: by subtracting the square, which is 100, from the given number, which is 300; the result it 200. It is known that 10 is the root of 100 and its double is 20. We cannot give it 10, because it would leave no number from which we could subtract the square of the quotient. Neither can we give it 9, because its square is too large, not even 8, because its square is also too large. So we give it 7. | ::You can extract the mentioned square by the first method I have mentioned for the square number 196: by subtracting the square, which is 100, from the given number, which is 300; the result it 200. It is known that 10 is the root of 100 and its double is 20. We cannot give it 10, because it would leave no number from which we could subtract the square of the quotient. Neither can we give it 9, because its square is too large, not even 8, because its square is also too large. So we give it 7. | ||
::<math>\scriptstyle{\color{blue}{\frac{300-10^2}{2\sdot10}=\frac{300-100}{20}=\frac{200}{20}=10>7\longrightarrow n=7}}</math> | ::<math>\scriptstyle{\color{blue}{\frac{300-10^2}{2\sdot10}=\frac{300-100}{20}=\frac{200}{20}=10>7\longrightarrow n=7}}</math> | ||
| − | |style="text-align:right;"|ותוכל להוציא המרובע הנז' על הדרך הראשונה שהזכרתי במרובע קצ"ו שתחסר המרובע שהוא ק' מהמספר הנתון שהוא ש' יהיה המספר ר' וידוע כי שרש ק' י' וכפלו כ'<br> | + | |style="text-align:right;"|ותוכל להוציא המרובע הנז' על הדרך הראשונה שהזכרתי במרובע קצ"ו שתחסר המרובע שהוא ק' מהמספר הנתון שהוא ש' יהיה המספר ר' וידוע כי שרש ק' י' וכפלו כ‫'<br> |
והנה לא נוכל לתת לו י' כי לא ישאר מספר שנוכל לחסר ממנו מרובע מה שיעלה בחלוק<br> | והנה לא נוכל לתת לו י' כי לא ישאר מספר שנוכל לחסר ממנו מרובע מה שיעלה בחלוק<br> | ||
| − | גם לא נוכל לתת לו ט' כי מרובעו גדול ואפי' ח' כי גם מרובעו גדול והנה נתן לו ז' | + | גם לא נוכל לתת לו ט' כי מרובעו גדול ואפי' ח' כי גם מרובעו גדול והנה נתן לו ז‫' |
|- | |- | ||
| | | | ||
| Line 5,989: | Line 7,781: | ||
| | | | ||
:*Example in the fourth rank: We wish to know the square that is closest to one-thousand and two-hundred. | :*Example in the fourth rank: We wish to know the square that is closest to one-thousand and two-hundred. | ||
| − | |style="text-align:right;"|<big>דמיון</big> במעלה הרביעית בקשנו לדעת המרובע הקרוב אל אלף ור' | + | |style="text-align:right;"|<big>דמיון</big> במעלה הרביעית בקשנו לדעת המרובע הקרוב אל אלף ור‫' |
|- | |- | ||
| | | | ||
| Line 5,995: | Line 7,787: | ||
::<math>\scriptstyle{\color{blue}{\frac{1200-30^2}{2\sdot30}=\frac{1200-900}{60}=\frac{300}{60}=5>4\longrightarrow n=4}}</math> | ::<math>\scriptstyle{\color{blue}{\frac{1200-30^2}{2\sdot30}=\frac{1200-900}{60}=\frac{300}{60}=5>4\longrightarrow n=4}}</math> | ||
|style="text-align:right;"|והנה בעבור שהוא מספר זוג נבקש במעלה השנית מספר דומה לזה והוא י"ב ומרובע שעבר הוא ט' וככה ט' מאות<br> | |style="text-align:right;"|והנה בעבור שהוא מספר זוג נבקש במעלה השנית מספר דומה לזה והוא י"ב ומרובע שעבר הוא ט' וככה ט' מאות<br> | ||
| − | וכאשר שרש ט' ג' ככה שרש ט' מאות ל'<br> | + | וכאשר שרש ט' ג' ככה שרש ט' מאות ל‫'<br> |
וכפלו ס' והנה נחסר המרובע שעבר מן החשבון הנתון והנה המרחק ג' מאות<br> | וכפלו ס' והנה נחסר המרובע שעבר מן החשבון הנתון והנה המרחק ג' מאות<br> | ||
| − | נחלקם על ס' והנה לא נוכל לתת לו ה' בעבור מרובע העולה בחלוק נתן לו ד' | + | נחלקם על ס' והנה לא נוכל לתת לו ה' בעבור מרובע העולה בחלוק נתן לו ד‫' |
|- | |- | ||
| | | | ||
| Line 6,051: | Line 7,843: | ||
::<math>\scriptstyle{\color{blue}{\frac{23000-100^2}{2\sdot100}=\frac{23000-10000}{200}=\frac{13000}{200}>50}}</math> | ::<math>\scriptstyle{\color{blue}{\frac{23000-100^2}{2\sdot100}=\frac{23000-10000}{200}=\frac{13000}{200}>50}}</math> | ||
|style="text-align:right;"|והנה זה דומה למעלה הראשונה כי איננו זוג והנה י' אלפים כמו אחד וכבר אמרנו מה שהוא במעלה הראשונה אחד יהיה במעלה החמישית מאה<br> | |style="text-align:right;"|והנה זה דומה למעלה הראשונה כי איננו זוג והנה י' אלפים כמו אחד וכבר אמרנו מה שהוא במעלה הראשונה אחד יהיה במעלה החמישית מאה<br> | ||
| − | והנה המרובע <ref>MS V530 begin.</ref>שעבר י' אלפים<br> | + | והנה המרובע ‫<ref>MS V530 begin.</ref>שעבר י' אלפים<br> |
נחסרנו מהמספר הנתון ישארו י"ג אלף<br> | נחסרנו מהמספר הנתון ישארו י"ג אלף<br> | ||
| − | נחלקנו על ר' שהוא כפל שרש המרובע הנמשל שעבר והנה נתן לו נ' | + | נחלקנו על ר' שהוא כפל שרש המרובע הנמשל שעבר והנה נתן לו נ‫' |
|- | |- | ||
| | | | ||
| Line 6,084: | Line 7,876: | ||
| | | | ||
::The addition is 400. We subtract it from the given number; 84600 remain and with the addition of 81, which is the square of the quotient, it is 84681, which is actually the square. | ::The addition is 400. We subtract it from the given number; 84600 remain and with the addition of 81, which is the square of the quotient, it is 84681, which is actually the square. | ||
| − | |style="text-align:right;"|והנה יש לו תוספת ת'<br> | + | |style="text-align:right;"|והנה יש לו תוספת ת‫'<br> |
נחסרם מהמספר הנתון ישארו פ"ד אלפים ות"ר ועם תוספת פ"א שהוא מרובע מה שעלה בחלוק יהיה פ"ד אלף תרפ"א וזה הוא המרובע באמת | נחסרם מהמספר הנתון ישארו פ"ד אלפים ות"ר ועם תוספת פ"א שהוא מרובע מה שעלה בחלוק יהיה פ"ד אלף תרפ"א וזה הוא המרובע באמת | ||
|- | |- | ||
| Line 6,101: | Line 7,893: | ||
::Since this rank is even, [the given number] is similar to twenty and the preceding square is 16. We have already said that what is units in the second rank is hundreds in the sixth rank, so the root is 400 and the preceding square is 160000. The difference is 40 thousand. We divide it by double the root, which is 800. We cannot give it 5 for the square of the quotient, so we give 4, which are 40. | ::Since this rank is even, [the given number] is similar to twenty and the preceding square is 16. We have already said that what is units in the second rank is hundreds in the sixth rank, so the root is 400 and the preceding square is 160000. The difference is 40 thousand. We divide it by double the root, which is 800. We cannot give it 5 for the square of the quotient, so we give 4, which are 40. | ||
::<math>\scriptstyle{\color{blue}{\frac{200000-400^2}{2\sdot400}=\frac{200000-160000}{800}=\frac{40000}{800}>40}}</math> | ::<math>\scriptstyle{\color{blue}{\frac{200000-400^2}{2\sdot400}=\frac{200000-160000}{800}=\frac{40000}{800}>40}}</math> | ||
| − | |style="text-align:right;"|ובעבור שזאת המעלה מן הזוגות היא דומה לעשרים והמרובע שעבר הוא י"ו וכבר אמרנו כי מה שהוא במעלה השנית אחדים יהיו במעלה הששית מאות והנה השרש ת' והמרובע שעבר ק"ס אלפים והנה המרחק מ' אלף נחלקנו על כפל השרש שהוא ת"ת והנה לא נוכל לתת לו ה' בעבור מרובע החלוק נתן לו ד' שהם מ' | + | |style="text-align:right;"|ובעבור שזאת המעלה מן הזוגות היא דומה לעשרים והמרובע שעבר הוא י"ו וכבר אמרנו כי מה שהוא במעלה השנית אחדים יהיו במעלה הששית מאות והנה השרש ת' והמרובע שעבר ק"ס אלפים והנה המרחק מ' אלף נחלקנו על כפל השרש שהוא ת"ת והנה לא נוכל לתת לו ה' בעבור מרובע החלוק נתן לו ד' שהם מ‫' |
|- | |- | ||
| | | | ||
| Line 6,166: | Line 7,958: | ||
נחסר ממנו מרובע מה שעלה בחלוק שהוא מ' אלף נשאר לנו ק"ס אלף<br> | נחסר ממנו מרובע מה שעלה בחלוק שהוא מ' אלף נשאר לנו ק"ס אלף<br> | ||
והנה שרש המספר שעבר אלפים ור' וכפלו ד' אלפים ות'<br> | והנה שרש המספר שעבר אלפים ור' וכפלו ד' אלפים ות'<br> | ||
| − | נחלק המספר הנשאר עליו נתן לו ל' | + | נחלק המספר הנשאר עליו נתן לו ל‫' |
|- | |- | ||
| colspan="2"| | | colspan="2"| | ||
| Line 6,174: | Line 7,966: | ||
::We are left with 28 thousand. We subtract from it the square of the quotient that is 30, which is 900; 27 thousand and 100 remain. The preceding root is two thousand and 230 and its double is 4 thousand and 460. We divide the remaining number by it; the result is 6. | ::We are left with 28 thousand. We subtract from it the square of the quotient that is 30, which is 900; 27 thousand and 100 remain. The preceding root is two thousand and 230 and its double is 4 thousand and 460. We divide the remaining number by it; the result is 6. | ||
|style="text-align:right;"|ישאר לנו כ"ח אלף<br> | |style="text-align:right;"|ישאר לנו כ"ח אלף<br> | ||
| − | נסיר ממנו מרובע מה שעלה בחלוק זה שהוא ל' והם תת"ק נשאר כ"ז אלף וק'<br> | + | נסיר ממנו מרובע מה שעלה בחלוק זה שהוא ל' והם תת"ק נשאר כ"ז אלף וק‫'<br> |
והנה השרש שעבר יהיה אלפים ור"ל וכפלו ד' אלפים ות"ס<br> | והנה השרש שעבר יהיה אלפים ור"ל וכפלו ד' אלפים ות"ס<br> | ||
| − | נחלק עליו המספר הנשאר יעלו ו' | + | נחלק עליו המספר הנשאר יעלו ו‫' |
|- | |- | ||
| colspan="2"| | | colspan="2"| | ||
| Line 6,201: | Line 7,993: | ||
::<math>\scriptstyle{\color{blue}{2000^2=4000000}}</math> | ::<math>\scriptstyle{\color{blue}{2000^2=4000000}}</math> | ||
::<math>\scriptstyle{\color{blue}{4999696-4000000=999696}}</math> | ::<math>\scriptstyle{\color{blue}{4999696-4000000=999696}}</math> | ||
| − | |style="text-align:right;"|כי כפל אלפים על אלפים יהיו שנים על שנים במעלה השביעית שהם ד' אלפי אלפים ונשאר לנו המספר הנז' | + | |style="text-align:right;"|כי כפל אלפים על אלפים יהיו שנים על שנים במעלה השביעית שהם ד' אלפי אלפים ונשאר לנו המספר הנז‫' |
|- | |- | ||
| | | | ||
| Line 6,222: | Line 8,014: | ||
::We multiply again two thousand twice by 6; the result is 24 thousand. | ::We multiply again two thousand twice by 6; the result is 24 thousand. | ||
::<math>\scriptstyle{\color{blue}{2\sdot2000\sdot6=24000}}</math> | ::<math>\scriptstyle{\color{blue}{2\sdot2000\sdot6=24000}}</math> | ||
| − | |style="text-align:right;"|נשוב לכפול אלפים על ו' פעמים<ref>MS V530 end</ref> יעלו כ"ד אלף | + | |style="text-align:right;"|נשוב לכפול אלפים על ו' פעמים‫<ref>MS V530 end</ref> יעלו כ"ד אלף |
|- | |- | ||
| | | | ||
| Line 6,260: | Line 8,052: | ||
::We multiply also 200 twice by 6; the result is two thousand and 400. | ::We multiply also 200 twice by 6; the result is two thousand and 400. | ||
::<math>\scriptstyle{\color{blue}{2\sdot200\sdot6=2400}}</math> | ::<math>\scriptstyle{\color{blue}{2\sdot200\sdot6=2400}}</math> | ||
| − | |style="text-align:right;"|ועוד נכפול ר' על ו' פעמ' יעלו אלפים ות' | + | |style="text-align:right;"|ועוד נכפול ר' על ו' פעמ' יעלו אלפים ות‫' |
|- | |- | ||
| | | | ||
| Line 6,273: | Line 8,065: | ||
| | | | ||
::We start with 30. | ::We start with 30. | ||
| − | |style="text-align:right;"|נחל מן ל' | + | |style="text-align:right;"|נחל מן ל‫' |
|- | |- | ||
| | | | ||
| Line 6,312: | Line 8,104: | ||
| | | | ||
:*Example: what is the ratio of 49 to 100? | :*Example: what is the ratio of 49 to 100? | ||
| − | |style="text-align:right;"|<big>דמיון</big> מה ערך מ"ט אל ק' | + | |style="text-align:right;"|<big>דמיון</big> מה ערך מ"ט אל ק‫' |
|- | |- | ||
| | | | ||
| Line 6,361: | Line 8,153: | ||
::We calculate its square: | ::We calculate its square: | ||
::*2 by 2 and 4 integers. | ::*2 by 2 and 4 integers. | ||
| − | |style="text-align:right;"|כפלנו מרובע זה והנה ב' על ב' ד' שלמי' | + | |style="text-align:right;"|כפלנו מרובע זה והנה ב' על ב' ד' שלמי‫' |
|- | |- | ||
| | | | ||
::*2 by 5 sevenths twice are 20 sevenths | ::*2 by 5 sevenths twice are 20 sevenths | ||
| − | |style="text-align:right;"|וב' על ה' שביעיות פעמי' הם כ' שביעיו' | + | |style="text-align:right;"|וב' על ה' שביעיות פעמי' הם כ' שביעיו‫' |
|- | |- | ||
| | | | ||
| Line 6,402: | Line 8,194: | ||
|style="text-align:right;"|<big>דמיון</big> חברנו ל"ו שהוא מרובע ו' עם ס"ד שהוא מרובע ח' ועם ד' מאות שהוא מרובע כ' והנה המחובר מג' מרובעי' הוא ת"ק<br> | |style="text-align:right;"|<big>דמיון</big> חברנו ל"ו שהוא מרובע ו' עם ס"ד שהוא מרובע ח' ועם ד' מאות שהוא מרובע כ' והנה המחובר מג' מרובעי' הוא ת"ק<br> | ||
כפלנוהו ג' פעמים עלו אלף ות"ק שמרנו זה החשבון<br> | כפלנוהו ג' פעמים עלו אלף ות"ק שמרנו זה החשבון<br> | ||
| − | נשוב לחפש היתרוני' והנה היתרון בין הו' ובין הח' ב' ומרובעו ד'<br> | + | נשוב לחפש היתרוני' והנה היתרון בין הו' ובין הח' ב' ומרובעו ד‫'<br> |
והיתרון בין הו' ובין הכ' י"ד ומרובעו קצ"ו<br> | והיתרון בין הו' ובין הכ' י"ד ומרובעו קצ"ו<br> | ||
היתרון בין הח' ובין הכ' י"ב ומרובעו קמ"ד<br> | היתרון בין הח' ובין הכ' י"ב ומרובעו קמ"ד<br> | ||
| Line 6,413: | Line 8,205: | ||
|- | |- | ||
|Likewise, if you sum 4 or 5 numbers and multiply them a certain number of times. | |Likewise, if you sum 4 or 5 numbers and multiply them a certain number of times. | ||
| − | |style="text-align:right;"|ועל זה הדרך אם חברת ד' מספרים או ה' ותכפלם כך פעמ' | + | |style="text-align:right;"|ועל זה הדרך אם חברת ד' מספרים או ה' ותכפלם כך פעמ‫' |
|- | |- | ||
|The rule is that you multiply by the number of squares that you sum, then always subtract the squares of the differences, as many as they are, from the sum; the remainder is a square. You just have to be sure to know the differences. | |The rule is that you multiply by the number of squares that you sum, then always subtract the squares of the differences, as many as they are, from the sum; the remainder is a square. You just have to be sure to know the differences. | ||
| Line 6,424: | Line 8,216: | ||
| | | | ||
:*Example: We sum 6 squares and we wish to know the number of the differences. | :*Example: We sum 6 squares and we wish to know the number of the differences. | ||
| − | |style="text-align:right;"|<big>דמיון</big> חברנו ו' מרובעי' ונרצה לדעת מספרי היתרוני' | + | |style="text-align:right;"|<big>דמיון</big> חברנו ו' מרובעי' ונרצה לדעת מספרי היתרוני‫' |
|- | |- | ||
| | | | ||
::We subtract one, as we have said; 5 remain. [The sum of] the numbers that precede it is 15, and so is number of the differences. | ::We subtract one, as we have said; 5 remain. [The sum of] the numbers that precede it is 15, and so is number of the differences. | ||
| − | |style="text-align:right;"|נחסר אחד כאשר דברנו ישארו ה' והמספרי' שהם לפניו יהיו ט"ו וככה יהיו מספרי היתרוני' | + | |style="text-align:right;"|נחסר אחד כאשר דברנו ישארו ה' והמספרי' שהם לפניו יהיו ט"ו וככה יהיו מספרי היתרוני‫' |
|- | |- | ||
| | | | ||
| Line 6,681: | Line 8,473: | ||
|style="text-align:right;"|דע כי לעולם יהיה בין ב' מספרים שהם על הסדר כמספר השנים שרשים | |style="text-align:right;"|דע כי לעולם יהיה בין ב' מספרים שהם על הסדר כמספר השנים שרשים | ||
|- | |- | ||
| − | |Look at the number you want: how much is its distance from the preceding square? If the difference between your number and the preceding square is as the root of the preceding square, it is the mean number. <math>\scriptstyle a^2+a</math> | + | |Look at the number you want: how much is its distance from the preceding square? If the difference between your number and the preceding square is as the root of the preceding square, it is the mean number. |
| + | :<math>\scriptstyle{\color{OliveGreen}{a^2+a}}</math> | ||
|style="text-align:right;"|והנה הסתכל במספר שתרצה כמה מרחקו מהמרבע שעבר אם היה המרחק בין מספרך ובין המרובע שעבר כשרש המרובע שעבר הוא מספר האמצעי | |style="text-align:right;"|והנה הסתכל במספר שתרצה כמה מרחקו מהמרבע שעבר אם היה המרחק בין מספרך ובין המרובע שעבר כשרש המרובע שעבר הוא מספר האמצעי | ||
|- | |- | ||
| − | |Extract any number that is less than the mean from the preceding square number <math>\scriptstyle a^2+b</math> | + | |Extract any number that is less than the mean from the preceding square number. |
| + | :<math>\scriptstyle{\color{OliveGreen}{a^2+b}}</math> | ||
|style="text-align:right;"|וכל מספר שיהיה פחות מהאמצעי הוציאהו מהמספר המרובע שעבר | |style="text-align:right;"|וכל מספר שיהיה פחות מהאמצעי הוציאהו מהמספר המרובע שעבר | ||
|- | |- | ||
| − | |If it is greater than the root of the preceding [square], extract it backwards from the succeeding square. <math>\scriptstyle a^2-b</math> | + | |If it is greater than the root of the preceding [square], extract it backwards from the succeeding square. |
| + | :<math>\scriptstyle{\color{OliveGreen}{a^2-b}}</math> | ||
|style="text-align:right;"|ואם היה יותר מהשרש שעבר הוציאהו אחורנית מהמרובע שלפניו | |style="text-align:right;"|ואם היה יותר מהשרש שעבר הוציאהו אחורנית מהמרובע שלפניו | ||
|- | |- | ||
| Line 7,701: | Line 9,496: | ||
== Notes == | == Notes == | ||
| − | + | ||
| − | + | <div style="text-align: right;"><div class="mw-collapsible mw-collapsed"><div class="mw-collapsible-content"> | |
| − | + | <br> | |
| − | + | <references group=note/> | |
| − | + | </div></div></div> | |
| − | + | ||
| − | |||
==Apparatus== | ==Apparatus== | ||
| − | + | ||
| − | + | ||
| − | + | <div class="mw-collapsible mw-collapsed"><div class="mw-collapsible-content"> | |
| − | + | <references /> | |
| − | + | </div></div> | |
| − | + | ||
| − | + | === Chapter One === | |
| − | + | ||
| − | + | <div style="text-align: right;"><div class="mw-collapsible mw-collapsed"><div class="mw-collapsible-content"> | |
| − | + | <br> | |
| − | + | <references group=I/> | |
| − | + | </div></div></div> | |
| − | + | ||
| − | + | === Chapter Two === | |
| − | + | ||
| − | + | <div style="text-align: right;"><div class="mw-collapsible mw-collapsed"><div class="mw-collapsible-content"> | |
| − | + | <br> | |
| − | + | <references group=II/> | |
| − | + | </div></div></div> | |
| − | + | ||
| − | + | === Chapter Three === | |
| − | + | ||
| − | + | <div style="text-align: right;"><div class="mw-collapsible mw-collapsed"><div class="mw-collapsible-content"> | |
| − | + | <br> | |
| − | + | <references group=III/> | |
| − | + | </div></div></div> | |
| − | + | ||
| − | + | === Chapter Four === | |
| − | + | ||
| − | + | <div style="text-align: right;"><div class="mw-collapsible mw-collapsed"><div class="mw-collapsible-content"> | |
| + | <br> | ||
| + | <references group=IV/> | ||
| + | </div></div></div> | ||
== Appendix I: Glossary of Terms == | == Appendix I: Glossary of Terms == | ||
Latest revision as of 09:36, 4 November 2024
Contents
- 1 Prologue
- 2 Chapter One – [Multiplication]
- 3 Chapter Two – Division
- 4 Chapter Three – Addition
- 5 Chapter Four – Subtraction
- 6 Chapter Five - Fractions
- 7 Chapter Six - Proportions
- 8 Chapter Seven - Extraction of Roots
- 9 Additional Excerpt
- 10 Notes
- 11 Apparatus
- 12 Appendix I: Glossary of Terms
- 13 Appendix II: Bibliography
| ראה ספר מחוקק באמונה | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| ותמצא בו לכל מספר תכונה | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| אשר חבר בנו מאיר למאיר | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| קטן שנים וחכם בתבונה[1] | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| ספר המספר[2] | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Prologue |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Numeration |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Since the sublime God alone has created in the upper world nine large heavenly spheres revolving around the earth, which is the lower world. | בעבור[note 1] כי השם הנשגב לבדו ברא בעולם העליון תשע עגולות גדולות סובבות את הארץ שהוא העולם השפל[3] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Reference to the triple repetition of the word sefer in Sefer Yezira [the Book of Creation] – interpretation of one of them as representing the concept of the nine numbers: | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| And the author of the Sefer Yezira has said that the paths of the wisdom are by Sefar and Sefer and Sipur and the Sefar is nine numbers. | ובעל ספר יצירה[note 2] אמר כי נתיבות החכמה הם בִּסְפָר[note 3] וְסֵפֶר[note 4] וְסִפּוּר[note 5] והנה הַסְּפָר תשעה מספרים[4] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כי תשעה סוף כל חשבון ואלה יקראו האחדים שהם במעלה הראשונה[5] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כי עשרה דומה לאחד ועשרים דומים לשנים שהם שני עשרות[6] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והיה ראוי שיקראוהו עֶשְׂרַיִם כאשר יקראו ממאה מאתים ומאלף אַלְפַּים רק בעבור חבריו הבאים אחריו שהם שלשים עד תשעים נהגו כמנהגם[7] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה שלשים מגזרת שלש וככה כלם[8] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה מאה דומה לאחד גם לעשרה ומאתים דומה לשנים גם לעשרים[9] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וככה אלף ורבבה שהם ראשי כללים למספרים הבאים אחריהם שהם א'י'ק' ב'כ'ר'[note 6][10] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והאות על זה[note 7][note 8] כשתעשה עגול[note 9] ותכתוב סביבו תשעה מספרים[11] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותכפול תשעה על עצמו והטעם להיותו מרובע ארכו כרחבו תראה זה וככה הוא[12] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה המרובע אחד ושמונים והנה האחד לשמאלו של תשעה שהוא ראש האחדים וח' שהוא כנגד שמונים בכלל לימין תשעה[13] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תכפול ט' על ח' יהיה המחובר ע"ב והנה ב' לשמאלו וז' שהוא כנגד ע' לימינו[14] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תכפול ט' על ז' יהיה המחובר ס"ג והנה ג' לשמאלו וו' לימינו שהוא כנגד ס'[15] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תכפול ט' על ו' יהיה המחובר נ"ד והנה ד' לשמאלו וה' שהוא כנגד נ' לימינו[16] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ובעבור כי חשבון חמשה הוא אמצעי בט' מספרים על כן נקרא חשבון עגול[note 10] כי הוא מתגלגל על עצמו[note 11] כי מרובעו יש בו חמשה[17] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר תכפול ט' על ה' יתגלגל הדבר בעגול כי האחדים יהיו לימין ט' והכללים לשמאלו כי המחובר הוא מ"ה והנה הה' מפאת ימין ט' והכללים לשמאלו שהוא ד' במקום המ'[18] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר תכפול ט' על ד' יהיה המחובר ל"ו והנה ג' כנגד שלשים[19] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר תכפול ט' על ג' יהיה המחובר כ"ז והנה ב' כנגד עשרים[20] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר תכפול ט' על ב' יהיה המחובר י"ח והנה א' כנגד עשרה[21] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Hence the checking scales are based on modulo 9 | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Therefore, the scales of a number that is multiplied by itself or by another is 9. | על כן מאזני מספר שהוא כפול על עצמו או על אחר הם ט'[22] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
The Positional Decimal System |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
על כן עשו חכמי הודו כל מספרם על תשעה ועשו צורות לט' מספרים ואני כתבתי במקומם א' ב' ג' ד' ה' ו' ז' ח' ט'[23] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה לעולם אם יש בידך מספר אחדים ותחלת הכללים שהם עשרות יכתוב בתחלה מספר האחדים ואחר כך מספר הכלל[24] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם אין לו מספר האחדים ויש לו מספר במעלה השנית שהם העשרות ישים כדמות גלגל[note 12] בראשונה להורות כי אין במעלה הראשונה מספר ויכתוב המספר שיש לו בעשרות אחריו[25] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם הכלל שלו מהמאות ומהעשרות יכתוב גלגל בראשונה ואחר כך מספר העשרות בשנית ומספר המאות בשלישית[26] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם יש לו מספר אלפים ברביעית ומספר עשרת אלפים בחמישית ומספר מאות אלפים בששית[27] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כי א'י'ק' יחזור ברביעית לאלפים [note 13][note 14] ובשביעית לאלף אלפים ובעשירית לאלף אלפי אלפים וככה עד אין קץ[28]
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם יש לו מספר אחדים ומאות ואין לו עשרות[note 15] יכתוב מספר האחדים בראשונה וגלגל בשנית ומספר המאה בשלישית[29] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועל זה הדרך ישים שנים גלגלים בראשונה או כפי מה שיצטרך עד אין חקר או באמצע[30] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Table of Contents |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| ואחר שהזכרתי זה אזכיר שערי זה הספר ונאמר שהם שבעה[31] | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
השער הא'[note 16] לכפול חשבון על עצמו[note 17] או על אחר[note 18] או כפל חשבון אחד על שנים חשבונות[note 19] או יותר או כפל חשבונות רבים על רבים[note 20][32] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
השער הב'[note 21] לחלק חשבון כלל על פרט או שנים כללים על פרט אחד או כללים גבוהים על כללים שפלים או כללים ופרטים על פרטים גם אדבר על המאזנים[note 22] של שער הכפל והחלוק[33] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
השער הג'[note 23] בחבור מספר על מספר[note 24] פרט עם כלל או כלל עם כלל[34] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
השער הד'[note 25] לחסר מספר ממספר[note 26] פרט מכלל או כלל מכלל גם אדבר על מאזני שער החבור והמגרעת[35] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| השער הה' על השברים והם על דרכים רבים שלמים על שלמים ונשברים עמהם או שלמים ונשברים עם שלמים ונשברים למיניהם או שברים עם שברים או שברים על שברי שברים או שברי שברים על שברי שברים בין לכפול בין לחלק בין לחבר בין לגרוע ומאזניהם[36] | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| השער הו' בערכים והוא שער נכבד מאד כי ממנו יוכל להוציא רובי השאלות הקשות ורוב הראיות מחכמת המזלות יצאו מזה הערך[37] | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| השער הז' על שרשי המרובעים והמאזנים שלהם כי הם רבים וחכמת המדות תלויה בשער הזה וזה השער חמור מכל השערים ואין כח במשכיל לדעת קדרות המאורות אם לא ילמד זה השער ויתרי קשתי העגול יצאו מהשער הזה[38] | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Chapter One – [Multiplication] |
השער הראשון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
Shortcuts |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כבר הזכרתי איך הם מעלות המספר והנה כשיבואו לך שנים מספרים לכפול כלל על כלל בין שיהיה על עצמו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| I will discuss the meaning of the foundation in my discussion about the secret of the one, with the help of God. | ועוד אדבר על טעם המוסד בדברי על סוד האחד בע"ה[I 2] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון רצינו לכפול שלשים על מאתים[I 3] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה דמיון שלשים שלשה ודמיון מאתים שנים כפלנו ב' על ג' והנה עלו ששה וזהו החשבון השמור | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון אחר בקשנו לכפול מאתים על שבע מאות[I 5] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה כפלנו שנים על שבעה והנה י"ד והוא השמור והנה מאתים מהמעלה השלישית וז' מאות גם כן מהמעלה השלישית נקח להם ששה ונחסר אחד הנה חמשה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועל זה הסדר תוכל לעשות עד אין קץ[I 7] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם היו שנים מספרים מרחקם מחשבון כלל כמרחק שנים מספרים אחרים רק האחד במגרעת והשני בתוספת דע כמה מרובע מספר הכלל וגרע ממנו לעולם מרובע החשבון היתר והחסר והנשאר הוא החשבון המבוקש[I 8] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון רצינו לכפול כ"ט על ל"א[note 27] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה חשבון הכלל הוא שלשים ומרובעו ט' מאות כי שלשה על שלשה הם תשעה [I 10]והיתרון והחסרון הוא אחד ומרובעו אחד חסרנום ממרובע הכלל והנשאר הוא המבוקש והוא תתצ"ט[I 11] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון אחר רצינו לכפול ס"ו על נ"ד[I 12] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה חשבון הכלל ס' והחסרון והיתרון הוא ששה והנה מרובע הכלל ג' אלפים ות"ר נחסר ממנו ל"ו שהוא מרובע החסרון והיתרון והנשאר הוא המבוקש[I 13] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון אחר המספר האחד ר"נ והמספר האחר ש"נ[I 14] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה הכלל הוא ש' ומרובעו צ' אלף נחסר ממנו מרובע נ' שהוא החסרון והיתרון ומספרו אלפים ות"ק והנשאר הוא המבוקש[I 15] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועל זה הסדר נוכל לעשות שאר המספרים הדומים לאלה שהחסרון כמו היתרון[I 16] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
[note 28]דרך אחרת נכבדת שהוצאתי בדרך השלישית שנקח שלישית החשבון ונדע כמה מרובעו ונקח כמוהו בכלל הגבוה[note 29] ממנו ונחסר מרובע השלישית ממנו והנשאר הוא המבוקש[note 30] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון בקשנו לדעת כמה מספר מרובע ג'[I 18] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נקח שלישיתו שהוא אחד ומרובעו אחד ועשרה שהוא הכלל הקרוב אליו נחסר ממנו מרובע אחד שהוא השלישית וישאר ט' והוא המבוקש[I 19] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון אחר בקשנו לדעת מרובע ט"ו[I 20] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ושלישיתו ה' ומרובעו כ"ה והדומה בכלל הקרוב אליו ר"נ חסר ממנו מרובע ה' שהוא השלישית ישאר רכ"ה[I 21] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון אחר בקשנו לדעת כמה מרובע כ"ד[I 22] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה שלישיתו ח' ומרובעו ס"ד ודמיונו במעלה הגבוהה ממנו תר"מ נחסר ממנו מרובע השלישית שהוא ס"ד ישאר תקע"ו והוא המבוקש[I 23] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם לא היה למספר שלישית שלמה ויהיה בו תוספת אחד חסר האחד מהמספר והוצא המספר המבוקש כמשפט שהראיתיך ומה שיעלה הוסף עליו המספר שיש לו שלישית והמספר בעצמו והמחובר הוא המבוקש[note 31][I 24] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון בקשנו לדעת מרובע ז' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה אין לו שלישית חסרנו ממנו אחד שהוא נוסף והנה שלישית הנשאר שנים ומרובעו ארבעה והנה בכלל הקרוב הדומה אליו מ' נחסר ממנו ד' שהוא מרובע השלישית וישאר ל"ו שהוא מרובע ו' נחבר אליו הו' שיש לו שלישית והז' שהיה מספרנו בראשונה ושניהם י"ג יהיה המחובר מ"ט והוא מרובע ז'[I 25] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון אחר רצינו לדעת כמה מרובע כ"ב[I 26] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה חסרנו אחד ונשאר כ"א ושלישיתו ז' ומרובעו מ"ט והנה בכלל הקרוב אליו ת"צ נחסר ממנו מ"ט שהוא מרובע השלישית נשארו תמ"א שהוא מרובע כ"א נוסיף כ"א גם כ"ב מחוברים שהם מ"ג יעלה המחובר תפ"ד וזהו מרובע כ"ב[I 27] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם היו שנים בין המספר שלנו ובין המספר שיש לו שלישית נעשה להפך שנוסיף על המספר שלנו אחד ונדע כמה מרובע מספר שיש לו שלישית ונחסר ממנו כמספר שיש לו שלישית וכמספר שהיה לנו והנשאר הוא המבוקש[I 28] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון בקשנו לדעת כמה מרובע כ"ג[I 29] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה בעבור שאין לו שלישית שלמה נוסיף אחד יהיו כ"ד ושלישיתו ח' ומרובעו ס"ד והדומה לו תר"מ נחסר ממנו ס"ד שהוא מרובע השלישית ישאר תקע"ו והוא מרובע כ"ד גם נחסר מזה המספר מ"ז שהוא כ"ד עם כ"ג מחוברים ויהיה הנשאר תקכ"ט והוא המבוקש[I 30] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The number of steps required for receiving the product: | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ודע כי אם יהיו שנים מספרים לכפול זה על זה יספיק לך פעם אחת[note 32] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם היה מספר אחד על שנים מספרים אתה צריך לעשות זה פעמים[note 33] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם על שלשה שלשה[I 33] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| And so on according to this rule. | ועל זה המשפט הכל[I 34] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם הם שני מספרים על שני מספרים אתה צריך לעשות זה ד' פעמים[I 35] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון רצינו לכפול י"ג על כ"ח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה כפלנו י' על כ' שהוא כלל גם י' על ח' עלו ר"פ ואחר כפלנו ג' על כ' גם על ח' עלו פ"ד והנה הכל שס"ד[I 36] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם היה כלל אחד כולל שני המספרים די לך בג' פעמים[I 37] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון בקשנו לכפול י"ג על י"ו[I 38] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה י' כולל שני המספרים והנה נחבר ג' עם ו' עם י' והנה יהיה מספרינו י"ט נכפול אותו בעשרה עלו ק"צ נכפול שני המספרים הקטנים שהם ג' על ו' יעלו י"ח והנה הכל ר"ח[I 39] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ויש שיספיק לך שני פעמים לבדם[I 40] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון בקשנו לכפול כ"ד על כ"ו[I 41] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה כ' כולל שני המספרים חברנו ד' עם כ"ו שהוא הגדול עלה המספר ל' כפלנו כ' על ל' עלו ת"ר וכפלנו הקטנים זה על זה עלו כ"ד והנה המבוקש תרכ"ד[I 42] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תכפול ג' מספרים על ג' אתה צריך לעשות זה ט' פעמים[I 43] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| According to this procedure for every number. | ועל זה הסדר כל החשבון[I 44] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| See, whether the number consists of one or many [digits]. | וראה אם המספר אחד או רבים[I 45] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Multiplication of evens and odds | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם הוא מספר זוג גם המחובר יהיה זוג[I 46] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וטעם הזוג הוא באחדים[I 47] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כי כל כלל הוא זוג[I 48] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם המספר האחד זוג והשני נפרד והטעם שאינו זוג איזה מהם שיהיה גם המחובר יהיה זוג[I 49] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם המספר האחד נפרד וגם כן האחר גם המחובר יהיה נפרד[I 50] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Written calculations |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Know that if the numbers to be multiplied by each other are many, you have to multiply them by writing the 9 characters that I have showed you. | ודע כי אם היו המספרים הנכפלים אלה על אלה רבים אתה צריך לכפול אותם במכתב ט' אותיות שהראיתיך[I 51] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והדרך הסלולה שתשים טורי המספר המעט עליונים ופירוש המעט בחשבון הכלל ולא תחוש מן הפרטים[I 52] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותשים בטור אחר שפל למטה החשבון שהוא כללו גדול | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם החשבון שכללו קטן הם יותר מספרים מחשבון שכללו גדול שים אותם עליונים ולא תחוש[I 53] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If you would do the other way around, it does no harm, just confuses the student a little. | ואלו היית עושה להפך לא יזיק רק יתבלבל מעט על התלמיד[I 54] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואחר שתשים המספרים כמה שיהיו בטור העליון והאחרים בטור השפל כפול הראשון של הטור העליון על הראשון שבטור השפל והעולה כתוב אותו כנגד הטור הראשון העליון[I 55] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואחר כן כפול המספר הראשון העליון על המספר השני השפל וכתוב בטור השלישי כנגד המספר השני העליון[I 56] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וככה לכל המספרים השפלים עם המספר העליון הראשון[I 57] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם כאשר תכפול הראשון העליון על שכנגדו בשפל ויתחבר במספר כלל ופרט תכתוב הפרט במקום הראוי לו והכלל במספרו תכתבנו בחשבון שהוא אחריו[I 58] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואחר שתשלים לכפול החשבון הראשון של הטור העליון על כל מספרי הטור השפל תחל לכפול המספר השני של הטור העליון על מספר הראשון של הטור השפל והעולה כתבהו בטור השלישי כנגד השני העליון[I 59] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואחר כן תכפול השני העליון על שני שבטור השפל ותכתבהו בטור השלישי במספר השלישי[note 34] שהוא שני למספר שהחלות עתה ממנו[I 60] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואחר כן תחל במספר השלישי העליון לכפול אותו על הראשון שבטור השפל והעולה תכתבהו כנגד טור השלישי שהחילות ממנו[I 61] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| This is the procedure for all endlessly with the rule that the units are into the lower rank and the decade following it in the next rank. | וככה המשפט לכלם עד אין קץ עם משפט הפרט להיות התחתון והכלל שיבא אחריו בטור השני לו[I 62] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If there is zero, whether in the top row or in the bottom row, the rule is to write it in its appropriate position, as is the rule for all numbers next to it. | ואם היה גלגל בין בטור העליון בין בטור השפל משפטו לכתבו במקום הראוי לו[note 35] כמשפט כל המספרים שעליו[I 63] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואחר כן תחל לחבר מה שעלה בטור העליון עם השפל[note 36][I 64] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם אין בו עשרות תכתוב מה שהוא בחבור[I 65] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם יש בו עשרה כתוב אחד אחריו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם יש בו יותר כתוב היותר מבחוץ בחבור שיש לך ובמקום העשרה כתוב אחד שני לו נוסף[I 66] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן תעשה לכל היוצאים מהטור העליון והשפל והוצא הנותר מעשרות מבחוץ[I 67] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואחר שידעת כמה הוא המחובר בטור השלישי ספור מעלותיו וראה אם היו כמספר מעלות השנים טורים העליונים ממנו בחסרון אחד תדע כי חשבונך אמת[note 37][I 68] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם המספר האחרון בטור העליון הנכפל במספר האחרון בטור השפל ממנו יוצא אל כלל[note 38] יהיה מספר מעלות הטור השלישי כמספר שני טורים העליונים בלי מגרעת אחד[I 69] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Check: casting out by 9 | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Finally, check it through the scales. | ובחן באחרונה במאזנים[I 70] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| You proceed as follows: | וככה תעשה[I 71] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Consider every digit in the top row in any rank as if it were units, sum them and cast out the nines from the sum if it is more than 9, or less than it write alone, and this is the scale of the top row. | חשוב כל חשבון שתמצא בטור העליון באיזו מעלה שיהיה כאילו הם אחדים[note 39] וחברם והוצא המחובר ט' ט' אם יותר ט' או פחות ממנו כתוב אותו לבדו והוא מאזני הטור העליון[I 72] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Do the same with the scale of the bottom row until you know how much its scale is. | ככה תעשה למאזני הטור השפל עד שתדע כמה המאזנים שלו[I 73] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Multiply the scale of the top row by the scale of the other row, then cast out the nines from the product and keep the remainder with you. | וכפול מאזני הטור העליון על מאזני הטור השני והנכפל הוציאהו ט' ט' והנשאר יהיה עמך שמור[I 74] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If the scale of one of the rows is 9, do not weary yourself to find the scale of the other row, because it will always be casted out by 9. | ואם מאזני אחד מהטורים יהיה ט' אל תיגע עצמך לבקש מאזני הטור האחר כי ט' יצא לעולם[I 75] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Then, examine the scale of the third row and see: if it is the same as the reserved, then your calculation is true, but if not, you were wrong. | ואחר בדוק מאזני הטור השלישי וראה אם היה שוה לשמור חשבונך אמת ואם לאו הנה טעית[I 76] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה רצינו לכפול קכ"ז על שנ"ה[I 77] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכתבנו קכ"ז בטור העליון כזה ומספר שנ"ה תחתיו אות אות במקומו כזה[I 78] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפלנו ז' על ה' עלו ל"ה כתבנו ה' במעלה הראשונה וג' שהוא ל' במעלה השניה[I 79] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד כפלנו ז' על ה' השני התחתון עלו ל"ה כתבנו ה' במעלה השנית תחת ג' וג' בשלישית[I 80] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד כפלנו ז' הראשון על ג' התחתון עלו כ"א כתבנו א' בשלישית תחת ג' וב' ברביעית[I 81] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד כפלנו ב' האמצעי העליון על ה' הראשון מן התחתון עלו י' כתבנו א' בשלישית תחת הא'[I 82] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד כפלנו ב' העליון על ה' השנית התחתון היו גם כן י' כתבנו א' תחת ב' ברביעית[I 83] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד כפלנו ב' העליון על ג' התחתון והיו ו' כתבנו אותו תחת א' ברביעית[I 84] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד כפלנו א' העליון האחרון על ה' הראשון התחתון עלו ה' כתבנוהו בשלישית[I 85] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד כפלנו א' על ה' השני התחתון עלו ה' כתבנוהו ברביעית[I 86] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד כפלנו א' העליון על ג' התחתון היו ג' כתבנוהו בחמישית אחר ב'[I 87] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה נשלם הכפל[I 88] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חברנו כל אלו המספרים כל מה שהוא ממעלה אחת יחד הכל ומה שיעלה יותר מעשרה או עשרה כתבהו אחר המעלה ההיא[I 89] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ויעלה המחובר מ"ה אלפים ופ"ה[I 90] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| P1050 marg. another method | דרך אחרת יותר קצרה עשויה בשליבה נק' בלשונ' ביריקוקלי ונעשית בזה הדרך שאין כותבי' רק האחדי' שבידך ושומרי' העשרו' לקבצם עם העשרו' מהמספר הבא אחריו וכן ממדרגה למדרגה עושין כן | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| דמ' תרצה לכפול קכ"ז על שנ"ה תכפול ז' על ה' עלה ל"ה כתו' ה' במדרגת האחדי' ושמור בפיך ובלבבך ל' שהם ג' במדרג' השנית וכפול ז' על ה' עלו ל"ה וג' עשרו' וג' עשרו' היו בידך בין הכל עלו ל"ח כתו' ח' במדרגה השנית ושמור ג' מאות | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| עו' תשוב תכפול ב' עם ה' עלו י' והיה ראוי לכותבו תחת העשרות בשורה אחרת תחת הראשונה ולפי שהו' ר"ל י' עשרות שהם מאה כתו' עגול במדרגת העשרו' ושמור אחד שהוא מאה וכפול ב' על ה' השני עלו י' וא' שהיה שמור הנה י"א כתו' א' במדרגת המאות ובידך שמור א' שהוא אלף | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| עוד כפול א' על וא' על ה' השני וכתו' במדרגת האלפי' ה' | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| ואח"כ קבץ הכל בדרך קבוץ וכתו' למטה כאשר אתה רואה | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Chapter Two – Division |
השער השני | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Introduction - Preliminary definitions |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Definition of a number: Know that that every number is a sum of units. | דע כי כל חשבון הוא חברת האחדים[II 1] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| One | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| One alone does not assume any change, no increase and no division. | והאחד לבדו לא יקבל שנוי [note 40] ולא רבוי [note 41] ולא חלוק [note 42]
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| It is the cause of every increase, change and division. | והוא סבת כל רבוי ושנוי וחלוק [note 43][II 3]
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| One is eternal. | והאחד קדמון לבדו [note 44]
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Every number created through it. | וכל חשבון מתחדש בעבורו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והוא יעשה בפאה אחת מה שיעשה כל חשבון בשתי פאותיו[II 4] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כי שנים לחשבון שלשה הפאה האחת שהיא לפניו וארבעה הפאה האחרת שהיא אחריו ושתי הפאות המחוברות ששה שהם כפל שלשה וככה כל מספר[II 5] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה האחד אין לפניו פאה ואחריו פאה אחת שהיא שנים והם כפל האחד[II 6] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Now I shall talk about every number that has whole integers without fractions. | ועתה אדבר על כל חשבון שיש לו אחדים שלמים בלי שבר[II 7] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Sexagesimal Fractions | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 12 zodiac signs: Know that the astrologers have divided the celestial sphere into twelve parts. | ודע כי חכמי המזלות חלקו את הגלגל על שנים עשר חלקים[II 8] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועשו זה בעבור ששנת השמש י"ב חדשי הלבנה ואין חשבון קטן מי"ב שיש לו חלקים רבים כמוהו כי יש לו אחדים שלמים בחציו ושלישיתו ורביעיתו וששיתו וחצי ששיתו[note 45][II 9][note 46] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| degrees: They divided each sign to thirty degrees. | וחלקו המזל לשלשים מעלות[II 10] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כי זה המספר יש לו אחדים שלמים יותר מי"ב כי יש לו חצי ושלישית וחמישית וששית ועשירית[note 47][II 11][note 48] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה עלה מספר מעלות הגלגל ש"ס[II 12] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה המספר יש לו חצי ושלישית ורביעית וחמישית וששית ושמינית ותשיעית ועשירית והנה לא יחסר לו רק השביעית[note 49][II 13] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר תכפול זה המספר על ז' יהיה העולה אלפים ותק"כ וזה החשבון כולל כל החלקים עד עשרה[II 14] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה חכמי המזלות כאשר יכפלו מעלות על מעלות יהיה המחובר מעלות שהם אחדים שלמים[II 15] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וככה כאשר יחלקו מעלות על מעלות יהיה העולה בחלוק מעלות שהם אחדים שלמים[II 16] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Written calculations |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Now I shall give you a rule how to divide every number, whether it consists of one, two or many digits: | ועתה אתן לך כלל איך תחלק כל חשבון בין שיהיה אחד או שנים או מספרים רבים[II 17] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Write them in one row, each according to its rank. | כתוב אותם בטור אחד כל אחד כפי מעלתו [note 50][II 18]
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Then, write the number by which you divide in another row, whether it consists of one or many digits, each according to its rank corresponding to each digit according to its rank in the top row, and leave a space between the upper row and the bottom row so that you can write a middle row in between them; whether the quotient consists of one or many digits, you place each one according to its rank. | ואחר כך כתוב החשבון שתחלק עליו בטור אחר בין שיהיה מספר אחד או רבים כל אחד כפי מעלתו ויהיה כל חשבון לפי מעלתו כנגד כל חשבון כפי מעלתו בטור העליון וריוח תשים בין הטור העליון והטור השפל כדי שתוכל לכתוב טור אמצעי ביניהם בין שיהיה העולה מספר אחד או מספרים רבים כל אחד תשים כפי מעלתו[II 19] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The number by which you divide should be less than the number that you divide by it. | וראוי להיות המספר שתחלק עליו פחות מהמספר המחולק ממנו וזה הדבר הוא בחלוק השלמים[note 51][II 20]
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| But this is only for the division of integers, not for fractions, as I will explain with the help of God. | ולא כן בשברים כאשר אפרש בעזרת האל[II 21] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| When you set up the rows, as I said, you start dividing by the last digit in the top row. | ובתקנך הטורים כאשר אמרתי תחל לחלק מהמספר האחרון שהוא בטור העליון[II 22] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Divide it by the last number in the bottom row. | ותחלק אותו על המספר האחרון שהוא בטור השפל[II 23] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Consider the two numbers, even though they are decades, consider them as units. | וחשוב שנים המספרים אע"פ שהם כללים חשוב אותם כמו אחדים[note 52][II 24] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The quotient: see how far the last digit of the bottom row is from the first digit, whether it is units or a zero, and as the number of the distance, return back. | והעולה בחלוק ראה כמה מרחק המספר האחרון מהטור השפל מהמספר הראשון בין שיהיו בו אחדים או גלגל וכפי מספר המרחק תשיב אחורנית [note 53][note 54][II 25]
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Write the quotient there above the bottom row, which is beneath the top row. | ושם תכתוב העולה בחלוק למעלה מהטור השפל שהוא למטה מהטור העליון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If a number that cannot be divided remains from the last digit, and [the division] has not yet reached the rank of the units, return the remaining number back to the preceding rank, which is lower than it. | ואם ישאר במספר האחרון חשבון שלא נתחלק [note 55] ולא הגיע למעלת האחדים [note 56] השב אחורנית המספר הנשאר למעלה הראשונה שהיא פחותה ממנו [note 57][II 26]
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Consider each unit as ten. | וחשוב כל אחד עשרה[II 27] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Then, divide by the number by which you divide, and write the quotient back from the first rank you wrote, before the first quotient. | ואחר כך חלק על המספר שחלקת עליו והעולה בחלוק תכתוב אותו אחורנית מהמעלה הראשונה שכתבת לפני מה שעלה בחלוק בראשונה[II 28] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Proceed always like this until you reach a number that is smaller than the divisor. | ככה תעשה תמיד עד שתגיע אל המספר שהוא פחות מהמחולק עליו[II 29] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Write the remainder above the top row according to its rank. | ואותו הנשאר תכתבנו למעלה מהטור העליון כפי מעלתו [note 58][II 30]
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| In the fifth chapter I will explain what you should do with it. | ובשער החמישי אפרש לך מה שתעשה ממנו[note 59][II 31] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון בקשנו לחלק ט' אלפים על ע'[II 32] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
בזאת הצורה[II 33] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה נשים ע' בטור השפל כפי מעלתו ונחשוב כי הכל אחדים והנה נתן לו א' [note 60][II 34]
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונכתבנו במעלה השנית אחורנית מהמספר האחרון שהוא בטור הראשון כי ע' הוא שני לטור השפל[note 61] ונכתבנו באמצע ונשארו לנו שנים[II 35] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נשיבם אחורנית והם עשרים נחלק על ז' והנה נתן לו ב' ונכתוב אותו אחורנית לפני הנכתב בראשונה[note 62] ונשארו לנו ו'[II 36] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נשיבהו אחורנית יהיו ששים נחלקנו על ז' נתן לו ח' תכתבהו אחורנית[note 63][II 37] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונשארו לנו ד' והם במעלה השנית והם מ' והמספר המחולק עליו גדול ממנו[II 38] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If the divisor as units is greater than the last digit in the top row, return it back and calculate from this position. | ואם היה המספר באחדים המחולק עליו גדול מהמספר האחרון בטור העליון תשיבהו אחורנית ותחשוב מאותו מקום [note 64][II 39]
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Return it back according to the distance of the divisor. | וכפי מרחק המספר המחולק עליו תשיב אחורנית [note 65][II 40]
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Proceed according to the rule. | ועשה כמשפט[II 41] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון בקשנו לחלק כ' אלף על צ'[II 42] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
בזאת הצורה[II 43] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה בעבור כי מספר ט' גדול מב' נשיבהו אחורנית והם כ' נחלקם על ט' והנה ב' נכתבנו במעלה השלישית אחורנית שהיא שנית לחשבון שחלקנו ממנו ונשארו ב'[II 44] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נשיבם אחורנית במעלה השלישית והם כ' נחלקם על ט' והנה ב' ונשארו ב'[II 45] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נשיבם אחורנית במעלה השנית והם עשרים נחלק על ט' והנם ב' ונשארו ב' שהם עשרים כי הם במעלה השנית בטור העליון וזה המספר פחות ממספרנו על כן נכתוב גלגל אחורנית כי לא עלו אחדים כי לא יצא לחוץ [note 66][II 46]
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If there is a zero in one of the positions and you cannot divide by the divisor, return it back from the higher [rank]. | ואם היה גלגל באחד המקומות ולא תוכל לחלק על המספר המחולק השב אחורנית מהגבוה ממנו[note 67][II 47] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון בקשנו לחלק ד' אלפים ול"ב על שלשים[II 48] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקנו ד' על ג' ועלה בידינו א' וכתבנוהו אחורנית במעלת המאות כי הוא שני לו נשאר לנו עוד א'[note 68][II 49] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נשיבהו אחורנית במעלת המאות[note 69] והיו י' נחלקנו על ג' ועלה בחלוק ג' נשאר לנו א'[II 50] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
השבנוהו אחורנית במעלת העשרות ויהיו י' חברנו אותו עם הכתוב במעלה השנית היו י"ג חלקנו אותו על ג' ונתנו לו ד' נשאר לנו א'[II 51] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
השיבונוהו אחורנית במעלה הראשונה היו י"ב שלא יתחלקו כי הנשאר פחות מאותו המחולק עליו וכבר יצא לחוץ[II 52] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If we want to divide one digit or two digits or many digits by one digit or two digits or three or many, provided that they are less than the digits in the top row, you proceed as follows: | וכאשר נרצה לחלק מספר אחד או שני מספרים או מספרים רבים על מספר אחד או על שני מספרים או על שלשה או על רבים על מנת שיהיו פחותים ממספרי הטור העליון ככה תעשה[II 53] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Give the last in the bottom row of the top row, as much as you can give it from the last digit in the top row. | תן לאחרון שבטור השפל מן הטור העליון מה שתוכל לתת לו מהמספר האחרון שבטור העליון[note 70][II 54] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Give the preceding in the bottom row, which is first to the last, as much as the product of the number you gave the last multiplied by the digit in the bottom row that precedes the last digit. | ותן לראשון מן הטור השפל שהוא ראשון לאחרון[note 71] ככפל המספר שנתת לאחרון על מספר הטור השפל שהוא לפני האחרון[note 72][II 55] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If you cannot do this, reduce the number that you gave it first. | ואם לא תוכל לעשות ככה שוב וגרע ממספרך שנתת לו בתחלה[note 73][II 56] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| When you have to take any digit from the digit that precedes the last, return it back as tens. | וכשאתה צריך לקחת מהטור שהוא לפני האחרון שום מספר השיבהו אחורנית לעשרות[note 74][II 57] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| This is how you proceed with all the ranks. | ככה תעשה לכל המעלות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If there is a zero in one of the ranks of the upper row, return back from the higher rank next to it as tens and take from them as much as is necessary. | ואם היה באחת ממעלות הטור העליון גלגל השב מן הגבוה ממנו אחורנית בעשרות וקח ממנו מה שצריך לו[note 75][II 58] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If there are two zeros in the ranks of the upper row and in the ranks of the lower row there are numbers, you return back the higher [number] that corresponds to the digit that follows the last zero and take what you need from it. Then you return the remainder back as tens, and take what you need for the multiple of the number that is in the bottom row from the rank from which it should be taken. | ואם היו שני גלגלים במעלות הטור העליון ובמעלות הטור השפל מספרים תשיב אחורנית הגבוה שהוא כנגד החשבון שהוא אחר הגלגל האחרון ותקח מהם מה שתצטרך ומהנשאר תשיב אחורנית בעשרות ותקח ממנו מה שתצטרך בכפל המספר שהוא בטור השפל מן המעלה שהיא ראויה לקחת ממנה[II 59] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה[II 60] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ד'ט' שכתבנו הוא הנשאר מן החשבון [II 61]שלא יתחלק[II 62] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה כאשר חלקנו ח' שהוא אחרון בטור העליון על ג' שהוא אחרון בטור השפל והנה נתנו לו שנים ובעבור שהיה הג' שבטור השפל שלישי החזרנו לשלישי ממנו אחורנית והגיע למעלת העשרות ונשאר לנו במספר הח' שנים[II 63] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה הנחנו שם אחד כי אחד יספיק לנו והחזרנו האחד אצל השנים והיו י"ב וחסרנו ממנו י' שהוא כפל חמשה האמצעי שבטור השפל והנה נשארו ב' על הב'[II 64] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נניח שם אחד ונחזיר אחד אחורנית על אחד שהוא שלישי ויהיו י"א נסיר ממנו ו' לג' שהוא ראשון שבטור השפל נשארו ה'[II 65] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה לכל הג' השפלים מה שראוי להם[II 66] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נשוב לחלק כי נשאר לנו א' על ח' וא' על ב' וה' על א'[II 67] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נשיב הא' שהוא על הח' אחורנית על א' אשר על הב' יהיו י"א נחלק אותם על ג' שהוא בטור השפל יהיו ג' ונכתבנו כנגד הטור הראשון שהוא לפני השנים ונשארו לנו ב'[II 68] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נשיבם על הה' אחורנית יהיו כ"ה נתן לחמשה האמצעי שבטור השפל ט"ו ונשארו עשרה[II 69] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נשיב אחד אחורנית על הג'[note 76] וישארו ט' והא' עם הג' י"ג ונקח מהם ט' וישארו ד'[II 70] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וט' על הה' כי לא יכולנו לקחת בראשונה הג' מהי' אע"פ שיספיק לו כי אינו מעלתו עכשיו אע"פ שלקח ממנו בראשונה כי בראשונה היה שלישי לו כי האחרון שבטור השפל לקח מן הח' אבל עתה לקח מן הב' א' ואחר שהוא שלישי צריך הוא שיקח ממעלתו השלישית[II 71] והנה הנשאר ד' ט'[II 72] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון אחר[II 73] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
באנו לחלק ט' על שנים והנה לא יכולנו לתת לו ד' כי לא ישאר אלא א' וכאשר תשיבהו אחורנית הנה עם הג' י"ג וט' ד' פעמים ל"ו על כן נתן לו ג' נשארו ג'[II 74] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נשיבם כלם אחורנית לשני לו שהוא ג' ויהיו שם ל"ג נתן לט' ג' יהיו כ"ז נשארו ו' על הג'[II 75] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נקח מהם א' ונניח ה' נשיבהו על הח' שהוא שלישי לאחרון שבטור העליון ועם הח' יהיו י"ח נתן אותם כלם לו' שהוא שלישי שבטור השפל נכתוב על הח' גלגל לפי שלא נשאר על הח' מאומה[II 76] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נשוב לחלק שעדין לא יצא לחוץ נקח מן הה' שהנחנו על הג' שהוא שני בטור העליון שנים שהוא אחד נכתוב זה האחד על הו' אחרי הג' ששמנו על הט' בחלוק הראשון והוא שלישי בטור השפל וכבר יצא לחוץ[II 77] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נקח מן הג' שעל הג' אחד וישארו שנים נתן האחד על הגלגל והם י' נתן לט' שבטור השפל ט' נשאר על הגלגל א'[II 78] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נשיבהו אחורנית על הא' שהוא רביעי וראשון בטור העליון והם י"א נתן לו ו' נשארו ה' על הא'[II 79] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה הנשארים על הטור העליון ה' וגלגל וב' שהם ר"ה ולא יתחלקו יותר שהג' שבטור השפל הם רצ"ו והנלקח לכל אחד ל"א[II 80] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון אחר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
בקשנו לחלק הטור העליון על הטור השפל לתת לו[note 77] ב' לא נוכל שלא ישאר כי אם א' וד' והם י"ד ויש לנו לחלק על ט'[note 78] ב' פעמים אך נתן לו א' ונשים אותו כנגד ט' שהם בטור העליון שהוא רביעי לה' אחורנית כשנים בטור השפל שהוא רביעי לה' ראש שבטור השפל נשארו ג' על הה'[II 81] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נקח מהם אחד ישארו ב' על הה' נשיבהו אחורנית על הד' יהיו י"ד יקח ט' ישארו ה'[II 82] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
יש לד' שבטור השפל לקחת מן השלישי שבטור העליון למען כי שלישי הוא ולא נוכל כי השלישי שבטור העליון גלגל הוא נשיב מן הה' שהנחנו על הד' אחד על הגלגל יהיו י' יקח ד' ישארו ו' על הגלגל[II 83] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
יקח הה' שהוא ראש בטור השפל והוא רביעי יקח מהרביעי שבטור העליון שהוא ט' ישארו על ט' ד'[II 84] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נשוב לחלק כי עדין לא יצא והנשארים ג' מן הטור העליון שהוא ראשון וחמישי ועל הט' ד' ועל הגלגל ו' ועל הד' ד' ועל הה' ב'[note 79][II 85] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נשיב הב' על הד' והם כ"ד נתן לב' שהוא רביעי שבטור השפל ח' נשארו ח' על הד'[II 86] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נשיב מהם ז' על הו' אחורנית וא' נשאר על הד' ויהיו ע"ו נקח לט' ע"ב נשארו ד' במקום הו'[II 87] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם נשיב מהם ג' ואמת כי הד' שבטור השפל יוכלו לקחת מהג' עם הד' שאחריהם שהם ל"ד אך לא ישאר כי אם שנים וכשנשיב אותו על הג' יהיו כ"ג לבד ויש לה' שיקחו מ'[II 88] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
לפיכך נשיב כל הד' אחורנית על הד' ונכתוב גלגל במקום הו' כנגד הגלגל שבטור העליון והם מ"ד יקחו הד' ל"ב נשארו י"ב[II 89] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נקח מהם ד' כי לא יהיה די לנו בפחות וישארו ח' על הד' שהם על הט' ועם הג' הם מ"ג יקחו הה' מ'[II 90] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ישארו ג' על הג' וח' על הט' שהם פ' וגלגל להוציאו ממאות ולהכניסו לאלפים וא' על הרביעי שהוא על הד' שהוא אלף ואלה לא יתחלקו כי המחולק גדול מזה שהוא אלפים וט' מאות ומ"ה[II 91] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון אחר בקשנו לחלק ס"ח אלפים וט' מאות וכ"א על ז' אלפים ונ"ג[II 92] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וזהו הדמיון[II 93] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ז' שהוא רביעי בטור השפל לא יוכל לקחת מו' שהוא חמישי בטור העליון ומאחר שלא נוכל לתת לז' כל הצורך[II 94] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נשיב אותו כלו אחורנית על הח' ויהיו ס"ח הנה חלקנו מן הח' שהוא רביעי לטור העליון נתן לז' ט' נוציאנו לחוץ על הג' שהוא רביעי בטור השפל נשארו ה' על הח'[II 95] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה השני לז' לא יקח מאומה כי הוא גלגל[II 96] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
יש לה' שיקח מן ב' הרביעי בטור העליון שהוא שלישי לחלוק לא יוכל לקחת[II 97] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נקח מן הט' שאחריו ה' נשיבם על הב' יהיו נ"ב וד' נשאר על הט' והה' מנ"ב יקחו מ"ה ישארו ז' על הב'[II 98] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
מן הז' נקח ג' ונשיבם אחורנית על א' והם ל"א יקחו ג' כ"ז נשאר ד' על א'[II 99] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון אחר נרצה לחלק תר"פ אלפים ות"ב על אלפים וט'[II 100] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
מספרים על מספרים שיהיה בטור העליון ב' גלגלים וכן בטור השפל[II 101] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נתן לב' הרביעי בטור השפל ג' מהו' הששי בטור העליון[II 102] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה הגלגל שני בטור השפל יש לו שיקח מן הח' ולא יקח כלום[II 103] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והגלגל השלישי שבטור השפל יש לו שיקח מן הגלגל השלישי בטור העליון ולא יוכל לקחת[II 104] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
יש לט' הראשון בטור השפל לקחת מד' שבטור העליון לא יוכל צריכין אנו שנשיב מן הח' השני בטור העליון מה שיספיק לו נשיב א' אחורנית כי די לנו בא' ונכתוב על הח' ז' והא' שהשיבונו אחורנית על הגלגל יצא לנו בעשרות ועוד לא יספיק נקח מהם ג' ונשיבם אחורנית ונשארו ז' על הגלגל והג' הם ל' על הד'[II 105] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נשארו ז' במקום ד' וגלגל ושנים הראשונים שבטור העליון וז' שעל הגלגל וז' שעל הח'[II 106] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד נשוב לחלק שהרי לא יצא נקח לו ג' מן הז' שעל הח' ונשימהו תחת הגלגל הראשון שהוא רביעי לח'[II 107] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונשאר א' על הח'[II 108] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
יש לגלגל שיקח מן הגלגל לא יוכל[II 109] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ויש לגלגל שאחריו שיקח ממעלתו שהיא הז' שעל הד' לא יוכל[II 110] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
יש לט' שיקח מן הגלגל שהוא מעלתו לפי שהוא רביעי לחלוק ולא יוכל לפי שאין על הגלגל כלום וגם לא יוכל להשיב אותו אחורנית על השנים כי השנים אינם מעלתו נשיב מן הז' שעל הד' שלפניו ג' נשימם על הגלגל והם ל'[II 111] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נשארו ג' על הגלגל וד' על הד' לפניו וז' על הגלגל שהוא לפני הח' וא' על הח'[II 112] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נשוב לחלק שעדין לא יצא לחוץ הנה מן הא' לא יוכל לקחת האחרון שבטור השפל נשיב אותו אחורנית על הז' שהוא על הגלגל והם י"ז נתן לו ח' נוציאם לחוץ אחרי הג' כי הוא רביעי לחלוק כי מן הז' שעל הגלגל חלקנו[II 113] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ושם נשאר א'[II 114] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה יש לגלגל שיקח מן הד' ולא יקח[II 115] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
גם יש לגלגל האחר שיקח מן הג' שעל הגלגל שבטור העליון ולא יקח[II 116] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ויש לט' שיקח מן הב' ולא יוכל נשיב אחורנית אם נאמר לג' שעל הגלגל שיתן לב' הראשון לגלגל אין לו מה שיספיק לו כי אין לו אלא ג' נקח מן הד' שבמקום הד' אחד ויהיה על הגלגל עם הג' י"ג נקח מהם ז' ונשיבם על הב' והם ע"ב יצאו הכל בט'[II 117] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונכתוב גלגל על הב' שלא נשאר עליו כלום ועל הגלגל שהוא שני לב' נשארו ו' וג' על ד' וא' על הגלגל שהוא שני לח' ואלה נשארו שלא יתחלקו כי המחולק גדול מזה כי המספר הנשאר אלף וג' מאות וס' והמחולק עליו הוא אלפים וט'[II 118] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון אחר נכבד וקשה מכל החשבונים שתחתיו מאין גלגל[II 119] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| First, I shall explain that whenever a digit is separated from a digit, meaning that a zero is written in between, as for example, 203, we do not say: we return the 2 or, as much is needed according to the digit in the lower row, back to the 3, because the zero is in the middle, so we return the 2 or the one back to the zero and then divide according to the rule. | ובראשונה אפרש כי לעולם כשיתרחק חשבון מחשבון והטעם שיכתב גלגל באמצע כדמיון זה ג0ב [II 120]לא נאמר נשיב הב' אל הג' או כמה שיצטרך לפי החשבון שבטור השפל לפי שגלגל באמצע אך נשיב הב' או האחד אל הגלגל ואז נחלק כמשפט[II 121] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| I shall tell you one rule for all the numbers you divide, whether they are many or a few: You always have to divide the upper number by the lower one, until the end of the digits when it has come to its end. If there is a zero above, it can no longer be divided. | ואומר לך כלל אחד מכל החשבונות שתחלק בין רבים בין מעטים לעולם יש לך לחלק חשבון העליון על התחתון עד שיצא לסוף החשבונות בא על סופו אם יש גלגל עליו לא יתחלק עוד[II 122] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כמו זה וזה צורת הנכבד והקשה[II 123] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון בקשנו לחלק חשבון ט' שביעיות על ד' תשיעיות[II 124][II 125] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה ראש כל דבר אוֹרְךָ איכה תחלק אחר שתראה שהז' פחות מהט'[II 126] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| First version | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תצטרך להשיבו אחורנית וזהו הדמיון ותחלה כשתחל לחלק תתן לט' ז' ותכתבנה אחורנית במעלת הד' שתחלק ממנו שהוא שני לט'[II 127] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנשאר י"ד וכן תעשה עד שישאר במעלת א' לחלוק ז' וכן בשני לחלוק ז' ובשלישי לחלוק ח' וברביעי לחלוק ד'[II 128] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נשוב לחלק נשיב הז' שהוא בשני אל השלישי אחורנית ונחלק ממנו ונכתוב ז' תחת ז' ששי שהוא ד' לחלוק והנשאר י"ד וכן תעשה עד שישאר ז' ואחריו נכתוב ח' ואחריו ה' ואחריו ד'[II 129] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נשוב לחלק נשיב ז' על הח' ונשים אותה במערכת ד' והנותר ט"ו ומה שתשאיר ח' ואחריו ה' ואחריו ה' ואחריו ד' ותכתוב ז' תחת השלישי שהוא הרביעי לחלוק[II 130] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נשוב לחלק נשיב ח' על ה' ונתן ח' תחת השמיני שהוא ז' רביעי לחלוק ישאר ה' על ה' ואחריו ה' ואחריו ה' ואחריו ה'[II 131] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נשוב לחלק נתן ה' על ה' אחורנית ונתן ה' תחת ז' התשיעי והוא רביעי לחלוק והנשאר י' ומה שתשאיר ה' ואחריו ה' ואחריו ו' ואחריו ב' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועוד לא יתחלק כי כבר יצא לחוץ בה' וכי הנותר הוא ה' אלפים וה' מאות וס"ב והמחולק עליו ט' אלפים וט' מאות וצ"ט והמקובל ע"ז אלף וז' מאות ופ"ה[II 133] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכלל חלוק החשבון ג' זיינין וג' חיתין וג' דלתין וט' ההין וו' אחת וב' אחת[II 134] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והכלל כי עם כל החלוקים ד' חוץ מן האחרון שנתמעט עד ג'[II 135] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Second version | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| לכן שימהו לאחור לז' ויהיו ע"ז וחלקם על ט' ולא תוכל ליתן לו יותר מז' לצורך שאר המספר וישארו י"ד תניח מהם ז' לחלוק השני וז' תשימהו לאחור על הז' השלישי ויהיו ע"ז וחלקם על ט' השני וישארו י"ד תניח מהם ז' לחלוק שני והז' תשימהו לאחור אל הרביעי יהיו ע"ז וחלקם על הט' השלישי וישארו י"ד תניח מהם ח' לחלוק שני והו' תשימהו לאחור אל הז' החמישי ויהיו ס"ז וחלקם על הט' הרביעי וישארו ד'[II 136] | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| נמצא שנשארו לחלוק השני ז' ז' ז' ז' ז' ח' ז' ז'[II 137] | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| החלוק השני תשלח ז' לאחור ויהיו ע"ז וחלקם על הט' הראשון וישארו י"ד תניח מהם ז' לחלוק שלישי והז' שלחהו לח' שלפניו ויהיו ע"ח וחלקם על הט' השני ותן לו ז' כמו כן וישארו ט"ו תניח מהם ח' לחלוק שלישי וז' תשלחהו לאחור אל הד' ויהיו ע"ד וחלקם על הט' השלישי וישארו י"א תניח מהם ה' לחלוק שלישי והו' שלחהו אל הז' ויהיו ס"ז וחלקם על הט' הרביעי וישארו ד'[II 138] | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| נמצא שנשארו לחלוק שלישי ז' ז' ז' ד' ה' ח' ז'[II 139] | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| החלוק השלישי תשיב ז' לאחור ויהיו ע"ח חלקם על ט' הראשון ותוכל לתת לו ז' ולא יותר וישארו ט"ו תניח מהם ח' לחלוק הרביעי והז' שימהו לאחור אל הה' ויהיו ע"ה וחלקם על ט' השני ותן לו ז' כמו כן וישארו י"ב תניח מהם ה' לחלוק רביעי והז' שימהו לאחור אל ד' ויהיו ע"ד וחלקם על ט' שלישי וישארו י"א תניח מהם ה' לחלוק הרביעי והו' שימהו אחורנית אל הז' ויהיו ס"ז וחלקם על הט' הרביעי וישארו ד'[II 140] | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| ונמצא שנשארו לחלוק רביעי ז' ז' ד' ה' ה' ח' | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| חלוק הרביעי שים ח' אחורנית על הה' ויהיו פ"ה תוכל לחלקם על הט' הראשון וליתן לו ח' וישארו י"ג תניח מהם ה' לחלוק חמישי והח' שלחהו אחורנית אל הה' ויהיו פ"ה וחלקם על הט' השני וישארו י"ג תניח מהם ה' לחלוק חמישי והח' שלחהו אחורנית אל הד' ויהיו פ"ד וחלקם על הט' השלישי ח' כמו כן וישארו י"ב תניח מהם ה' לחלוק חמישי והז' שלחהו לאחור אל הז' ויהיו ע"ז וחלקם על הט' הרביעי וישארו ה'[II 141] | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| ונמצא הנשאר לחלוק חמישי ז' ה' ה' ה' ה' | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| חלוק חמישי שים ה' אחורנית ויהיו נ"ה תן מהם ה' על הט' הראשון וישארו י' תניח מהם ה' בלתי מחולקים והה' תשלח אחורנית אל הה' השלישי ויהיו נ"ה ותן על הט' השני ה' כמו כן וישארו י' תניח מהם ה' בלתי מחולקים והה' שלחהו אל ה' הרביעי ויהיו נ"ה ותן על הט' השלישי ה' כמו כן וישארו י' תניח מהם ו' בלתי מחולקים והד' שלחהו אל הז' ויהיו מ"ז וחלקם על הט' הרביעי ה' כמו כן[II 142] | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| וישארו בלתי מחולקים ב' ו' ה' ה'[II 143] | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Check | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| We complete by knowing the test for whether you divided correctly. | והנה נשלים לדעת המאזנים אם חלקת נכונה[II 144] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דע מאזני המספר שחלקת עליו בין שיהיה אחד או רבים[II 145] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
גם דע מאזני המספר שעלה בחלוק שכתבת בין שני הטורים בין שיהיה אחד או רבים[II 146] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכפול זה על זה האמצעי על התחתון ודע כמה נשאר על ט' ט' והוא השמור אם לא נשאר לך מספר שנשאר שהוא פחות מהמספר שחלקת עליו[II 147] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כי אם נשאר קח המאזנים שלו וחבר אותו עם השמור שהיה לך והמחובר הוא השמור באמת[II 148] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וראה מאזני המספר הגדול שחלקת אותו שהיה בטור העליון אם היה[II 149] שוה למאזני השמור תדע כי חשבונך אמת[II 150] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תכפול מה שיעלה בחלוק על המספר שחלקת עליו אחר שתחבר אליהם מה שנשאר לחלק אז יהיה המחובר שוה למספרי הטור העליון וחלוק נכון[II 151] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Additional excerpts[II 152] | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The rule of Division | כלל החלוק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If the number you by which you divide [= the divisor] is two digits or more, divide the last of the top row by the last of the bottom row, if the digit of the top row is greater than that of the bottom [row]. | אם המספר שתחלק עליו שני מספרים או יותר חלק סוף הטור העליון על סוף הטור השפל אם מספר העליון גדול מהשפל[II 153] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| But if the lower one is greater than the upper one, return the last of the upper one back as tens to the preceding rank and add it to what is written there. | ואם השפל גדול מהעליון השב סוף העליון לעשרות אחורנית על המעלה הקודמת לה ותצרפם עם הנכתב בה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If there is a zero in the preceding rank, count the tens. | ואם במעלה הקודמת לה גלגל ספור העשרות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Take from there what you can give the last lower digit. Write down what you can give to all the other bottom digits, as what you give to the last, and write the quotient in the middle between the two rows, far from the rank you divided as the distance from the last bottom digit from the starting digit. | וקח ממנו מה שתוכל לתת למספר השפל האחרון וכתוב מה שתוכל לתת לכל מספרי השפל האחרים מה שתתן לאחרון והיוצא כתבהו באמצע שני הטורים רחוק מן המעלה שחלקת ממנה כמרחק סוף השפל מראשו[II 154] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Proceed like this for all the divisions, doing the same for them as for this division: count from the rank which you divided as the distance of the last bottom digit from its starting digit, and write the quotient there. Then, from the digit that is second to the last bottom digit take the digit that is next to the rank from which you divided, as much times as the number that is written between the rows. | וכן תעשה לכל החלוקים שתשוב עליהם בזה החלוק שתמנה מן המעלה שחלקת ממנה כמספר סוף השפל מראשו ושם תכתוב היוצא בחלוק ומהמספר השני לסוף השפל תקחנו מן הסמוך למעלה שחלקת ממנו כמספר הכתוב באמצע הטורים[II 155] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If it is not enough for you, return what is left of the rank that you first divided back to the preceding rank and add it to what is written in it, then take your number from it. Do this with all. | ואם לא יספיק לך תשיב מה שנשאר מן המעלה שחלקת תחלה אחורנית אל המעלה הקודמת לה ותצרף עם מה שנכתב בה וקח ממנה מספרך וכן תעשה לכלם[II 156] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון רצינו לחלק פ"ג אלפים ותקכ"א על תתק"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה הט' שבטור השפל יותר מח' שהוא סוף העליון על כן תשיב הח' אחורנית על הג' יהיו פ"ג ונתן לט' מהפ"ג ט' יהיו פ"א ונשארו ב' על הג'[II 157] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והגלגל לא יוכל לקחת מן הה' העליון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והג' התחתון לא יוכל לקחת מן הה' העליון כי אינו מעלתו לכן השיב מן הה' שלשה על הב' יהיו ל"ב נסיר מהם כ"ז שהם ט' פעמים ג' שהוא ראשון השפל ונשארו ה' על הב' וב' על הה'[II 158] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ובעבור שהט' התחתון הוא במעלה השלישית וכבר יצא בחלוק ט' נכתבנו במעלה השנית שהוא שלישי לג' העליון שחלקת ממנו[II 159] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נשוב לחלק ונשיב ב' אשר על הג' אל הב' אשר על הה' יהיו כ"ב נסיר מהם ב' פעמים ט' כי ט' הוא השפל ונשארו ד' על הה' ונכתוב ב' במעלה הראשונה שהיא שלישית לה' העליון שחלקת ממנו בזה החלוק[II 160] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה הגלגל לא יוכל לקחת מאומה מן הה' אשר על הב'[II 161] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והג' השפל לא יוכל לקחת מן הא' העליון ב' כי אין בו רק אחד על כן נשיב אחד מן הה' שעל הב' אל הא' יהיו י"א ונקח מהם לג' התחתון ו' שהם ב' פעמים ג' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונשארו ד' על הה' וד' על הב' וה' על א' שלא יתחלקו[II 162] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון אחר רצינו לחלק י"א אלף וש"נ על ק"י[II 163] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
לקחנו א' מן הא' האחרון העליון וכתבנוהו במעלה השלישית תחת הג' העליון לפי שא' האחרון השפל הוא במעלה השלישית וככה נרחיק היוצא מסוף המספר שהחלות לחלק ממנו[II 164] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן קח מא' הרביעי העליון א' השני התחתון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ובעבור שלא נשאר במעלה הרביעית מאומה ונצטרך לשוב לחלק מהג' העליון נקח מהם ג' לא' האחרון מהשפל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונכתוב ג' במעלה הראשונה שהיא שלישית לג' העליון שהחלנו עתה לחלק ממנו ולכן שמנו גלגל במעלה השנית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה כי בחלוק הראשון החלונו מסוף העליון ולכן כתבנו היוצא בחלוק ברחוק ג' מעלות ממנו אחורנית כמספר מעלות סוף השפל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אבל בחלוק השני הזה החלונו לחלק מהג' העליון ולכן נכתוב היוצא רחוק ג' מעלות אחורנית והגיע זה אל המעלה הראשונה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וא' השני בטור השפל נקח מה' העליון ישארו ב' על הה' והם עשרים שלא יתחלקו[II 165] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה היוצא בחלוק ק"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| from here proceeds to the check | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Chapter Three – Addition |
השער השלישי[III 1] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Sums |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| It is written in the books of the arithmeticians that he who wants to know how much is the sum of the numbers that proceed successively up to a known number, multiplies it by its half plus one half and the result is the sum.
|
כתוב בספרי חכמי החשבון כי הרוצה לדעת כמה המחובר מן המספרים שיעברו על הסדר עד סוף מספר ידוע יכפול אותו על חציו בתוספת חצי אחד והעולה הוא המחובר[III 2] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון רצינו לדעת כמה הם המספרים המחוברים מא' עד סוף י"א[III 3] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה ידענו כי חצי י"א ה' וחצי נוסיף חצי אחד הנה יהיו ו' והנה נכפול י"א על ו' יעלו ס"ו והוא המחובר[III 4] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון אחר בזוגות כמה המחובר עד סוף י"ח[III 5] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה לקחנו חציו והוא ט' כפלנו י"ח עליו ועלו קס"ב ועוד יש לכפול חשבון י"ח על חצי אחד יעלו ט' חבר אותו עם קס"ב יעלו קע"א והוא המחובר[III 6] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Every number is in accordance with these two ways. | ועל אלו שני הדרכים הולך כל חשבון[III 7] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Another way: add one to the last number, multiply by half the number and the result is the sum.
|
דרך אחרת הוסף על סוף המספר אחד שלם וכפול על החצי מהמספר והעולה הוא המחובר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון בנפרדים כמה המחובר עד סוף י"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הוספנו אחד והיו י"ב והנה חצי י"א ה' וחצי כפלנו י"ב על ה' וחצי עלו ס"ו וככה המחובר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ובזוגות עד סוף י"ח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה חציו הוא ט' הוספנו אחד על י"ח היו י"ט כפלנו י"ט על ט' עלו קע"א[III 8] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Abraham the author said: | אמר אברהם המחבר [III 9]
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| I have found another way: add to the square of the last number the root that is the last number itself, then see how much is the sum and half the sum is the required.
|
מצאתי דרך אחרת תוסיף על מרובע סוף החשבון השרש שהוא בעצמו וראה כמה המחובר וחצי המחובר הוא המבוקש[III 10] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון ידענו כי מרובע י"א קכ"א ואחר נוסיף על י"א שהוא השרש והוא סוף החשבון יעלו קל"ב וחציו ס"ו[III 11] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| From this way you can derive all the questions that are concerning this matter. | ומזה הדרך תוכל להוציא כל השאלות שהם בענין הזה[III 12] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון שאל שואל חברתי מספרים עד שהגיעו למספר ידוע ועלה המחובר תס"ה כמה הוא סוף החשבון[III 13] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Always double the sum, then take the root of the preceding square and check it: if between the square and the double remains the root no more and no less, know that the calculation is correct and the [last] number is itself the root.
|
כפול לעולם החשבון המחובר וקח שרש הנכפל שעבר ובחון אותו כי אם נשאר בין המרובע ובין הנכפל כמו השרש בלי תוספת ומגרעת תדע כי החשבון נכון והחשבון בעצמו השרש[III 14] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה כפלנו תס"ה ועלה תתק"ל וידוע כי המרובע שעבר היה תת"ק ושרשו ל' והוא סוף המספרים המחוברים והנה אין בין המרובע והנכפל כי אם ל' והוא סוף החשבון[III 15] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Another general way for an even and an odd number [of terms]: double the square of half the [last] number, then add to it the root of this square, which is half the number and the result is the required.
|
דרך אחרת כוללת לזוגות ולנפרדים תכפול מרובע חצי המספר ותוסיף עליו שורש זה המרובע שהוא חצי החשבון והעולה הוא המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון רצינו לדעת כמה המחברים עד י"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה חצי המספר ו' והמרובע ל"ו כפלהו יהיו ע"ב הוסיף על זה ו' שהוא השורש עלו ע"ח והוא המבוקש[III 16] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Another question: we sum the squares up to a known number, how much is the sum?
|
שאלה אחרת חברנו כל המרובעים שהם עד סוף החשבון שהוא ידוע כמה המחובר[III 17] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| You should know the sum of the numbers preceding the [last] mentioned number including [the last number] and we name it and call it a sum, then we take two thirds of the [last] mentioned number plus one third, multiply it by the sum and the result is the required, which is the sum of the squares up to the mentioned number.
|
יש לך לדעת אותו החשבון שהזכיר כמה יעלה המחובר מהמספרים שלפניו ועמו נשים שם לאותו המספר הידוע ונקראנו סכום והנה נקח שתי שלישיות המספר שהזכיר עם תוספת שלישית אחד ונכפול זה המחובר על סכום המספר והעולה הוא המבוקש והוא המחובר מהמרובעים עד סוף המספר הנזכר[III 18] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון רצינו לדעת כמה המרובעים שהם עד סוף שבעה[III 19] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכבר ידענו שהמחובר כ"ח ונשוב לדעת כמה שתי שלישיות שבעה עם תוספת שלישית אחד והם חמשה כי שתי שלישיות ששה הם ארבעה ויש לנו עוד שתי שלישיות ועם תוספת שלישית אחד והנה נכפול על הסכום ה' ועלו ק"מ והוא המבוקש[III 20] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון אחר כמה המחובר מהמרובעים שהם עד סוף י"ב[III 21] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וידוע כי הסכום שלו ע"ח ושתי שלישיותיו ח' נכפלנו על ע"ח יעלו תרכ"ד נחבר אליו שלישית הסכום שהוא כ"ו יעלו תר"נ והוא המבוקש[III 22] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| From this way you can derive all the questions that are concerning this matter. | ועל זה הדרך תוכל להוציא כל השאלות שהם מזה הענין[III 23] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Additional excerpts | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| I have found an easy way to sum the cubes: | ואני מצאתי דרך נקלה לחבור המעוקבים[III 24] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If you want to know the [sum of the] cubes up to a known number, know the sum of the numbers up to that last number and take its square - it is the sum of the cubes up to the last number.
|
שאם תרצה לידע המעוקבים שהם עד מספר ידוע דע המספר המחובר עד סוף המספר ההוא וקח מרובעו והוא יהיה חבור המעוקבים עד סוף החשבון ההוא[III 25] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון רצינו לידע כמה מספר המעוקבים המחוברים עד ה' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה מספר המחובר עד סוף ה' הוא ט"ו קח מרובעו יהיו רכ"ה והוא יהיה מספר המעוקבים עד ה'[III 26] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Another matter: to know the sum of all the doubles.
|
ענין אחר לדעת כמה המחובר מכל הנכפל[III 27] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| It is [equal to] double the last minus the first.
|
והוא כפל האחרון במגרעת הראשון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון כי המחובר מן ג' ו' י"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הם כ"ד בחסרון ג' הרי כ"א והוא המחובר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון אחר א' ב' ד' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הם ח' במגרע א' הרי ז' והוא המחובר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Likewise, if one says only the last number. | וכן אם לא יגיד כי אם סכום אחרון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כמו אם ישאל כמה הם הכפולים זה על זה עד ל"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפול ל"ב יהיה ס"ד תחסר אחד שהוא ראשון ישאר ס"ג והוא המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| תמצא חבורו מראשו כמ' שהראיתיך עתה ויהיו כ"ח ושמרהו ועוד תקח מן הז' שני שלישיתיו ושליש והם ה' וכפול ה' בכ"ח ששמרת ויעלו | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| אז תדע חבורו מראשו ועד י"ב והם כמ' שידעת ע"ח ושמרהו וחזור ותקח שני שלישי י"ב והם ח' ותכפלם על ע"ח יהיו תרכ"ד ועוד חבר לאלו שליש ע"ח והם | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| ודע כי החבור הוא שתחל לחבר האחדים תחלה ואחר כן העשרות וכן בסדר וזה הפך החלוק שהוא יעשה אחורנית | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| דרך אחרת קח המחובר מן י"ב והם ע"ח ותקח שני שלישי י"ג והם ח' וב' שלישים והסר מהם שליש אחד וישארו ח' ושליש ותכפלם על ע"ח תדע כי הם יעלו כמו כן תר"נ | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם יאמר כמה המחובר מהמרובעים עד ח' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| קח חבורו של ח' והם ל"ו ובקש שני שלישי ט' והם ו' ותכפלם בל"ו ויעלו רי"ו הסר מהם שליש ל"ו והם י"ב וישאר ר"ד והוא המבוקש | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שאלה כמה הוא המחובר מד' עד ט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| אז תעשה כמ' שלמדתיך למטה לכפול ה' בט' ויעלו מ"ה והסר מהם ב' פעמים ג' והם ו' וישארו ל"ט והוא המבוקש | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|
ואם עוד ישאל כמה היא המחובר מהמרובעים כסדר מד' ועד ט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| אז תעשה כמ' שצויתיך למעלה למצוא מחוברם מראשם והם מ"ה ושמרם וקח שני שלישי ט' והם ו' וכפלם במ"ה ויעלו ר"ע ועוד שליש מ"ה והם ט"ו ויעלו רפ"ה ותסיר מזה מרובע א' ב' ג' והם י"ד וישארו רע"א והוא המבוקש | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| ועל הדרך הזה תוכל להוציא כל השאלות מזה הענין[III 28] | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| There is no need to mention all of this, but I shall only mention the method of the scales. | ואין צורך להזכיר כל זה רק אזכיר דרך המאזנים[III 29] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Written calculations |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| When you add a number to a number, even if they [consist of] many [digits], put one number in the upper row according to its ranks, put also the other number according to its ranks in the lower row. | כשתחבר מספר אל מספר בין שיהיו רבים אלו ואלו שים המספר האחד בטור העליון כפי מעלותיו גם שים המספר השני כפי מעלותיו בטור השפל[III 30] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Then add each [digit] in its rank and write the sum in a third row. | אחר כן חבר כל אחד אל מעלתו והמחובר כתוב אותו בטור שלישי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Then add the scale of the top row to the scale of the bottom row. | אחר כן חבר מאזני הטור העליון אל מאזני הטור התחתון[III 31] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If the sum of the two is equal to the scale of the third row, know that your calculation is correct. | ואם היה העולה בין שניהם כמאזני הטור השלישי אז תדע כי חשבונך אמת[III 32] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Now I shall explain how to approach the tables of astrology and how to take add seconds to minutes and minutes to degrees and degrees to signs. | ועתה אפרש היאך יכנס בלוחות חכמת המזלות והיאך יצרף שניים לראשונים וראשונים למעלות ומעלות למזלות[III 33] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Addition of Sexagesimal Fractions |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Now I shall give you a general method of astrology. | ועתה אתן לך דרך כוללת בחכמת המזלות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The celestial sphere was divided into 12 signs and the sign into 30 degrees. | חלקו הגלגל על י"ב מזלות והמזל על ל' מעלות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The degrees are as units in the number; each degree is divided into sixtieths, which are called minutes. | והמעלות הם כמו אחדים במספר וכל מעלה מתחלקת על ששים יקראו ראשונים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Each minute is also divided into sixtieths, which are called seconds. | גם כל ראשון יתחלק לששים עוד ויקראו שניים[III 34] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| There are no more fractions than these in the tables of the planets. | ואין בלוחות המשרתים שברים יותר מאלה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Know that the tables of the planets of the mean motion are according to two ways: | ודע כי לוחות המשרתים במהלך האמצעי על שני דרכים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
האחד על שנות השמש והם שנות הכלל מחוברים עשרים עשרים[III 35] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והדרך השנית על שנות הלבנה ושנות הכלל מחוברים שלשים שלשים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| I will explain this in the book | ובספר טעמי הלוחות אפרש זה[III 36] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| When they want to know the position of any planet required at any hour required, they approach the years of the decades that have passed and write down the number of the signs they find written there, and they write this at the beginning of a row. | והנה ברצותם לדעת מקום איזה משרת שיבקשו באיזו שעה שירצו יכנסו בשנות הכלל שעברו ויכתבו מה שימצאו כתוב שם ממספר המזלות ויכתבו זה בתחלת הטור[III 37] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Then, they write the degrees they find, after the first number in the same row and they do a long dividing line between the two numbers. | ואחר יכתבו מה שימצאו במעלות [39]אחרי המספר הראשון באותו הטור בעצמו וישימו הפרש בין שני המספרים בקו ארוך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Then, they write the minutes they want find after the degrees and the signs and make a separation between the two numbers with a long line in the same row. | אחר כן יכתבו מה שימצאו מן הראשונים אחרי המעלות והמזלות וישימו הפרש בין שני המספרים בקו ארוך באותו הטור בעצמו[III 38] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Then, they write the seconds after the minutes in the same row and separate between them with a long line. | אחר כן ישימו השניים אחרי הראשונים באותו הטור בעצמו ויפרישו ביניהם בקו ארוך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Then, they approach the single years that have passed and write what they find, the signs under the signs, the degrees under the degrees, the minutes under the minutes and the seconds under the seconds. | ואחר כן יכנסו בשנות הפרט שעברו ויכתבו מה שימצאו במזלות תחת המזלות והמעלות תחת המעלות והראשונים תחת הראשונים והשניים תחת השניים[III 39] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Then, they approach the months that have passed and write everything they find there, each type under its type. | ואחר כן יכנסו כנגד החדשים שעברו ויכתבו כל מה שימצאו שם כל מין תחת מינו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Then, they approach the days of the month that have passed of a month that has not been completed and write everything they find there each type under its type. | ואחר כן יכנסו בימות החדש שעברו אל החדש שלא נשלם ויכתבו כל מה שימצאו כנגדם כל מין תחת מינו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The same is done with the whole hours that have passed after the middle of the day and the same with the parts of an hour that has not been completed. | וככה יעשו בשעות השלמות שעברו אחר חצי היום וככה בחלקי השעה שלא נשלמה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Then, one starts to add up all the sixtieths: takes one minute for every sixty seconds and write how many minutes come out of the seconds with the minutes that precede the seconds, and write the remainder of the seconds that are less than sixty alone in another place. | ואחר כן יחל לחבר כל הששים ויקח לכל ששים שניים חלק ראשון ויכתוב כמה ראשונים יעלו מן השניים עם הראשונים שהם לפני השניים והנשאר מן השניים שהם פחותים מן הששים יכתבם לבדד במקום אחר[III 40] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Then, one adds again all the minutes: takes one degree for every sixty minutes and write the degrees that are summed with the degrees that precede the minutes and the remaining minutes that are less than sixty, write them in the row where you wrote the seconds, only that they are written before the seconds. | ואחר כן ישוב לחבר כל הראשונים ויקח לכל ששים ראשונים מעלה אחת ומה שיתחבר מן המעלות כתוב אותם עם המעלות שהיו לפני הראשונים והראשונים הנשארים שהם פחותים מששים כתוב אותם בטור שכתבת שם השניים רק יהיו נכתבים לפני השניים[III 41] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Then, add again the degrees: take one sign for every thirty degrees and write the [signs] that come out with all the [signs] that you had and what remains of the degrees that are less than thirty, write them alone before the minutes that you wrote before the seconds. | ואחר כן שוב לחבר המעלות וקח לכל שלשים מעלות מזל אחד וכתוב המעלות העולים עם כל המעלות שהיו לך ומה שישארו מן המעלות שהם פחותים משלשים כתוב אותם לפני הראשונים שכתבת לפני השניים לבדד[III 42] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Then, take out every twelve signs you find, and write the remainder alone before the degrees you wrote before the minutes preceding the seconds. | ואחר כן הוצא כל שנים עשר מזלות שתמצא והנשאר כתוב אותם לפני המעלות שכתבת לפני הראשונים שכתבת לפני השניים לבדד[III 43] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| So, you have the position of the planet on the celestial sphere along with its degrees, minutes and seconds. | אז יהיה לך מקום המשרת בגלגל המזלות עם מעלותיו וחלקיו ושנייו[III 44] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| You always start to count the signs from the sign of Aries. | ולעולם תחל לספור המזלות מראש מזל טלה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If the number of signs is twelve, write a zero in the place where the signs must be written to indicate that the planet is still in the sign of Aries, for this is not yet complete, but that it has only moved away from the sign in accordance with the degrees that have been written there and the minutes belong to the following degree. | ואם היה מספר המזלות שנים עשר כתוב אותם במקום הראוי להכתב שם מזלות גלגל להודיע כי המשרת עודנו במזל טלה כי לא נשלם רק עבר מן המזל כפי המעלות שתמצא כתובות ויהיו הראשונים מן המעלה הבאה[III 45] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כי אם היו המעלות י"ז יהיו הראשונים הם שעברו ממעלת י"ח ונחשוב כי הראשונים השלמים היו ט"ו שלמים ונאמר כי השניים היו מ"ה והנה הם שלש רביעיות החלק הראשון של ששה עשר ראשונים[III 46] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Chapter Four – Subtraction |
השער הרביעי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Written calculations |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Subtracting one number from the another is easy. | לגרוע חשבון אחד מחשבון אחר קל הוא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| I shall give you only a general method of subtracting many digits from many digits, using the method of the arithmeticians as well as the method of the astrologers, for they have a different method. | רק אתן לך דרך כוללת לגרוע חשבונים רבים מחשבונים רבים על דרך חכמי החשבון גם על דרך חכמי המזלות כי דרך אחרת יש להם[IV 1] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Do as follows: | וככה תעשה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Write the number from which you want to subtract [= the minuend] in the upper row and write the digits you want to subtract [= the subtrahend] in the lower row. | כתוב החשבון שתרצה לגרוע ממנו בטור העליון וכתוב המספרים שתחפוץ לגרעם בטור השפל[IV 2] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The last digit in the top row must always be greater than the last digit in the bottom row. Do not consider the other digits. | ולעולם יהיה המספר האחרון בטור העליון גדול מהמספר האחרון שהוא בטור השפל ואל תחוש מן המספרים האחרים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If you find in one of the ranks that the digit in the lower row is greater than the corresponding digit in the upper row, return one alone from the digit that follows, for that is enough for you and consider it as ten, as the way we do in the division. | והנה אם מצאת באחת מן המעלות[note 80] מספר הטור השפל גדול מהמספר שבטור העליון שהוא כנגדו השב אחורנית מהמספר שאחריו אחד לבדו כי יספיק לך וחשוב אותו עשרה על הדרך שאנו עושין בחלוק[IV 3] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון הטור העליון ב'ג'ד'ה' והטור השפל ט'ז'ג'ב' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וראוי לגרוע כל אחד מהשפלים מן העליונים כל אחד ממעלתו ב' מה' וג' מד'[IV 4] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולא נוכל לחסר ז' מג' ולא ט' מב'[IV 5] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| We always start to subtract backwards, as with the way of division, and write the remainder in the third row corresponding to the same rank in the lower row. | ולעולם נחל לחסר אחורנית[40] כדרך החלוק [note 81] והנשאר נכתבנו בטור השלישי כנגד אותה המעלה שבטור השפל[IV 6]
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה גרענו ב' מה' נשאר לנו ג' כתבנו אותו תחת מעלה הרביעית[IV 7] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חסרנו ג' מד' נשאר לנו אחד ולא כתבנוהו רק שמנו גלגל במקומו כי הוצרכנו להשיב אחד אחורנית כי המספר שבטור השפל לפניו גדול משכנגדו העליון והנה היו י"ג חסרנו ממנו ז' ונשארו ו' רק בעבור כי החשבון הראשון שבטור השפל גדול מן העליון שבטור העליון על כן הוצרכנו להשיב אחורנית אחד ונכתוב ה' בטור השלישי והנה היו למעלה י"ב נחסר ט' ונשארו ג'[IV 8] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וזו היא הצורה[IV 9] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| When you want to know the scales: | וכאשר תרצה לדעת המאזנים[IV 10] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Subtract the scale of the lower row from the scales of the upper row and keep the remainder. | תגרע מאזני הטור השפל ממאזני הטור העליון ותשמור הנשאר[IV 11] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| See: if the scale of the third row is the same, then you know that your calculation is correct. | ותראה אם היו מאזני הטור השלישי כמוהו דע כי חשבונך אמת[IV 12] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If the scale of the bottom row is greater than the scale of the top row, always add 9 to the scale of the top, subtract the scale of the bottom from the sum and proceed according to the instructions. | ואם היו מאזני הטור השפל גדול ממאזני הטור העליון הוסף לעולם על מאזני העליון ט' וגרע מהמחובר מאזני השפל ותעשה כמשפט[IV 13] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Example. We subtract 1 from 2; 1 remains. We return it back to the zero; they are 10. We subtract 7; 3 remain. | דמיון החסרנו א' מב' נשאר א' השיבונו אחורנית על הגלגל והיו י' חסרנו ז' נשארו ג'[IV 14] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The scale of the lower [row] is 8 and the scale of the upper [row] is 2. We add 9 to it; they are 11. We subtract 8; 3 remain and so is the bottom scale. | והנה מאזני השפל ח' ומאזני העליון ב' הוספנו עליו ט' והיו י"א החסרנו ח' נשארו ג' וככה מאזני השפל[IV 15] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Now, we shall speak about the method of the astrologers, because they need this chapter more than the arithmeticians. | עתה נדבר על דרך חכמי[41] המזלות כי יותר צורך יש להם לשער הזה מחכמי החשבון[IV 16] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| We already mentioned that you should set up the signs in the first rank, degrees in the second, the minutes in the third and seconds in the fourth. | וכבר הזכרנו כי כן תכון המזלות במעלה הראשונה והמעלות בשנית והראשונים בשלישית[42] והשניים ברביעית[IV 17] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| One always starts to subtract backwards: the seconds in the bottom row from the seconds in the top row. The remainder is written in the third row corresponding to the upper seconds. | ולעולם יחלו לגרוע אחורנית השניים בטור השפל מהשניים בטור העליון והנשאר יכתבהו בטור השלישי כנגד השניים העליונים[IV 18] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If the lower seconds are more than the upper ones, he takes one from the upper minutes, considers it as 60 seconds, adds to them the seconds that are in the upper row, and then subtracts the lower seconds according to the instructions. | ואם היו השניים השפלים רבים מהעליונים יקח מהראשונים העליונים אחד יחשבהו ס' שניים ויחבר אליהם השניים הנמצאים בטור העליון ואחר כן יגרע השניים השפלים כמשפט[IV 19] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If he has taken one from the upper [minutes], he subtracts it from the number of minutes that are there. | ואם לקח אחד מהעליונים יגרענו מהמספרים הראשונים שהיו שם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Then, he subtracts the lower minutes from the upper minutes that are there and writes the remainder corresponding to them in the third row. | ואחר כן יחסר הראשונים השפלים מהראשונים העליונים הנמצאים שם ויכתוב הנשארים כנגדם בטור השלישי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Then, he subtracts the degrees from the degrees and write the [remainder] corresponding to them in the third row. | ואחר כן יחסר מעלות ממעלות והנשארים יכתבם בטור השלישי כנגדם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If the degrees in the lower row are more than the degrees in the upper row, he takes one from the signs, considers it as 30, adds them to the degrees that are written there, then subtracts, but makes sure that he subtracts one from the number of the signs that are written in the first [rank]. | ואם היו המעלות בטור השפל רבות ממעלות הטור העליון יקח מהמזלות אחד ויחשבנו ל' ויחברם אל המעלות הכתובות שם ואחר כך יגרע וישמר שיגרע אחד ממספר המזלות הכתובים בראשונה[IV 20] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Then, he subtracts the signs from the signs and write the remainder in the third row corresponding to them. | ואחר כך יגרע מזלות ממזלות ויכתוב הנשארים בטור השלישי כנגדם[IV 21] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If the lower signs are more than the upper ones, he always adds 12 to the upper ones, then subtracts and proceeds according to the instructions. | ואם היו המזלות השפלים גדולים מהעליונים יוסיף לעולם על העליונים י"ב ואחר כן יגרע ויעשה כמשפט[IV 22] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| To know the scales: | לדעת המאזנים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| One starts with the seconds, subtracts the scale of the lower seconds from the scale of the upper ones, keeps the remainder and see if the scale of seconds in the third row is just as much, then his calculation is correct. | יחל מהשניים ויגרע מאזני השניים השפלים ממאזני השניים העליונים וישמור הנשאר ויראה אם היה כמוהו מאזני השניים בטור השלישי חשבונו אמת[IV 23] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If he sees that he has taken one minute from the minutes and added to the seconds, he adds 6 to the scale of the upper seconds and then subtracts according to the instructions. | ואם ראה שלקח ראשון אחד מן הראשונים ושם עם השניים יוסיף על מאזני השניים העליונים ו'[note 82] ואחר כך יגרע כמשפט[IV 24] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| He does with the scales of the minutes as he has done with the seconds. | ויעשה למאזני הראשונים כאשר עשה לשניים[IV 25] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If he had to take a degree and convert it to 60 minutes to add them to those that are written there, add 6 to the scale of the minutes that are there, then subtract and proceed according to the instructions. | ואם הוצרך לקחת מעלה והשיבה לס' ראשונים לחברם עם הכתובים שם הוסף על מאזני הראשונים שהיו שם ו' ואחר כך תגרע ותעשה כמשפט[IV 26] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Do with the scales of the degrees the same as with the minutes and seconds. | גם כן תעשה במאזני המעלות כמשפט הראשונים והשניים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If you have taken from the signs one that you have set with the degrees, add 3 to the scale of the degrees that are written, then subtract and proceed according to the instructions. | ואם לקחת מן המזלות אחד ששמת אותו עם המעלות הוסף על מאזני המעלות הכתובים בראשונה ג' ואחר כן תגרע ותעשה כמשפט[IV 27] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Do with the scales of signs the same as you did with all of them. | ועשה במאזני המזלות כמשפט שעשית בכל אלה[IV 28] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If you added 12 to the original signs, add 3 to the scale of the signs that were first written, then subtract and proceed according to the instructions. | ואם הוספת על המזלות הראשונים י"ב הוסף על מאזני המזלות הכתובים בראשונה ג' ואחר כך תגרע ותעשה כמשפט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| I shall tell you a rule, one thing that is necessary when subtracting: | כלל אומר לך דבר שהוא צורך למגרעת[IV 29] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The last [digit] at the end of the upper row must always be greater than the last [digit] in the lower row, when the rows are the same, the upper [has] the same [number of ranks] as the lower one. | לעולם האחרון שבסוף הטור העליון יהיה גדול מהאחרון שבטור השפל כשהטורים שוים שהעליון כמו התחתון[IV 30] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If the rows are not the same, as the upper one is greater than the lower one in respect of the number of digits, as here [igure is missing], return one back and that is enough. | ואם אין הטורים שוים שהעליון גדול מן התחתון במספר אותיות כזה ישיב האחד אחורנית ודי לו[IV 31] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| דרך אחרת לגרוע חשבון מחשבון בהפך שכתוב למעלה שיחל לגרוע מן האחדים[IV 32] | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| דמיון רצינו לגרוע ט' מב' והנו כזו הצורה | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| נקח מג' שלפניו ויחזירנה לאחור ויהיו י"ב נשארו ג' ויכתבם בטור השלישי | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| ועתה נרצה לגרוע ז' מג' ולא נוכל כי לא נשאר במקום הג' כי אם ב' נקח מן הד' א' ונחזירהו לאחור על הג' שהוא ב' ויהיו י"ב גרע מהם ז' וישארו ה' ונכתבנו בטור השלישי כנגד הז' | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| ואחר כן נגרע ג' מד' שהוא ג' שכבר חסרנו ממנו א' ויצא זה כנגד זה לכן נכתוב גלגל בטור השלישי תחת הג' | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| ואחר כן נגרע מה' וישארו ג' ונכתבנה בטור השלישי תחת הב' | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Chapter Five - Fractions |
השער הה' הוא שער השברים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| It is known that the one is as a point in a circle; therefore one cannot be a fraction. | ידוע כי האחד כמו נקודה בתוך עגולה [43] על כן לא יתכן להיות האחד נשבר
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The whole is named with one name, as the shape represents the entire body. | רק בעבור שיקרא הכלל בשם אחד כמו הצורה שהיא כוללת כל הגוף
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The body is composed of surfaces. | והגוף מורכב משטחים [44]
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Therefore man considers the one in thought as fractions and fraction of fractions . | ובעבור זה יעשה האדם מן האחד שברים במחשבה ושברי שברים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The arithmeticians take all their fractions from a great number so that its fractions are whole units. | וחכמי החשבון יקחו כל שבריהם מחשבון גדול שיהיו שבריו אחדים שלמים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Thus, they take the half from two. | על כן יוציאו החצי משנים
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The third from three. | והשליש משלשה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| And so on to the end of the first rank of the number. | וככה עד סוף המערכת הראשונה בחשבון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The analogous number from which they derive is called the "denominator". | והדמיון שיקחו ממנו יקראוהו המורה
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| For the product is divided by its square. | כי על מרובעו יחלקו העולה בחשבון
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The remainder that cannot be divided is one part of it or a number of parts that can be named by the name of the units, such as one-quarter, one-third and their similar. | והנשאר שלא יתחלק יהיה חלק ממנו או חלקים שיוכל להזכירו בשם האחדים כמו רביעית שלישית והדומה להם
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Sometimes the denominator is a number that has no parts that man can express, because it is a prime number that is not composed, such as 11 or 13, and their like. | ויש פעמים שיהיה המורה חשבון שאין לו חלקים שיוכל האדם לבטא בהם כי הוא חשבון ראשון איננו מורכב כמו י"א או י"ג והדומים להם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| I have already mentioned that the first rank consists of 9 numbers. | וכבר הזכרתי כי המערכת הראשונה ט' מספרים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The one, on the one hand, is not a number, and on the other hand, it is a number. | והנה האחד מפאה אחד איננו מספר ומפאה אחרת הוא מספר
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| It is similar to an odd number: | והוא דומה לנפרד [45]
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Because when you sum all the odd numbers together successively the squares are generated. | כי בחברך כל הנפרדים זה על זה על הסדר יולדו המרובעים
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| As well as many other matters, which there is no need to mention. | ודברים רבים אין צורך להזכירם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| So, eight numbers remain in the first rank, half of which are prime numbers, and the other half are composite numbers. | והנה נשארו במערכת הראשונה שמנה מספרים והנה חציים ראשונים וחציים מורכבים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הראשונים הם שנים שלשה חמשה ושבעה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והמורכבים ארבעה ששה שמנה ותשעה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| When there is a need for two fractions that are not of one kind, which are not similar to each other, one looks for each of the fractions: from which number each of them is derived and multiplies one number by the other; the product is the denominator. | וכאשר יצטרכו לשנים שברים שאינם ממין אחד שלם שלא ידמה זה לזה יבקשו כל אחד מהשברים מאיזה חשבון יצא כל אחד מהם ויכפלו חשבון האחד על האחר והעולה בחבור הוא המורה
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If there are 3 types, one multiplies the number from which the [first] fraction is derived by the second number from which the second fraction is derived and multiply the product by the number from which the third fraction is derived. | ואם היה ג' מינים יכפול החשבון שיצאו ממנו השברים על החשבון השני שיצאו ממנו שברי השני והמחובר יכפלהו על המספר שיצאו ממנו שברי השלישי
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The same is done if there are 4 types or more: one looks for a common denominator for all. | וככה יעשה אם היו ד' מינים או יותר כי יבקשו מורה אחד לכלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| It is called by this name [moreh, lit. indicator], because it indicates the straight path; if you want, name it by a different name, it does no harm. | ונקרא בשם הזה בעבור כי הוא יורה הדרך הישר ואם תרצה קרא לו שם אחר כי לא יזיק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| After I have told you the methods of the denominator, I will tell you how you can calculate two fractions of two denominators, for it is in a shorter way. | ואחר שאומר לך דרכי זה המורה אומר לך איך תוכל להוציא שני שברים משני מורים כי הם יותר דרך קצרה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| After I will finish talking about the fractions in the method of the arithmeticians and their argumentations, I will explain you the fractions of the astrologers, for they have a different method. | ואחר שאשלים לדבר על שברי דרך חכמי החשבון ומחלוקותיהם אפרש לך שברי חכמי המזלות כי דרך אחרת להם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| I will start giving you examples from the easy ones, then I will mention the difficult ones. | ואחל לתת לך דמיונות מן הקלים ואח"כ אזכיר הכבדים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| First, I shall tell you a rule: that the multiplication of fractions is opposite to the multiplication of integers. | ואומר לך כלל בתחלה כי כפלי השברים הפך כפלי השלמים
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Because when it is said: multiply one-half by one-half it is as if it is said: take one-half of one-half; the result is one-quarter. | כי האומר כפול חצי על חצי כאלו אומר קח חצי החצי והנה העולה רביעית אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| We know that the half is derived from two; its half is 1 and the other half is also one; one multiplied by one is one; the square of the denominator is 4, so this one is one-quarter, that is the half of one-half. | ידענו כי החצי יצא משנים והנה חציו אחד וחצי האחר גם הוא אחד והנה אחד על אחד אחד והנה מרובע המורה ד' והנה זה האחד הוא רביעי והוא חצי החצי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| We proceed oppositely to our practice with the integers, because we are always looking for the ratio of the product to the square of the denominator. | והנה נעשה להפך מנהגנו בשלמים כי נבקש לעולם מהו ערך הנכפל ממרובע המורה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכפל שלישית על שלישית יהיה העולה תשיעית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכפל רביעית על רביעית יהיה העולה י"ו והנכפל אחד והנה הוא חצי שמינית [46]
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| In this way up to ten and beyond. | ועל זה הדרך עד עשרה וככה למעלה ממנו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כמו חלק אחד מי"א כפול על חלק אחד מי"א והנה חלק אחד מקכ"א שהוא המרובע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| In this way you multiply fractions of one type by fractions of the same type, whether they are the equal or one of them is greater than the other, then you divide the product by the square of the denominator. | ועל זה הדרך תכפול שברי מין האחד על שברי המין בעצמו בין שיהיו שוים או שיהיה אחד מהם גדול מהאחר ואח"כ תחלק על מרובע המורה הנכפל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון רצינו לכפול ג' רביעיות על ג' רביעיות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה המורה ד' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
לקחנו לכל אחד מג' רביעיות ג' והנה הנכפל ט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקנו אותם על י"ו שהוא מרובע המורה הנה הוא חציו וחצי שמיניתו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תרצה חלק ט' על ד' והדבר יצא שוה כי רביעית הרביעית חצי השמינית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון בקשנו לכפול ג' חמישיות על ד' חמישיות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה המורה ה' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
לקחנו בעבור הג' חמישיות ג' ובעבור הד' חמישיות ד' כפלנו ד' על ג' עלו י"ב והוא הנכפל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה ב' חמישיות המרובע וב' חמישיות חמישית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If one says fractions of two kinds: | ואם אמר שברים מב' מינים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שיאמר כפול לי ב' שלישיות אחד על ג' רביעיותיו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נבקש המורה לשניהם ונכפול ג' על ד' והוא המורה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה נקח בעבור ב' השלישיות ח' וג' רביעיות ט' נכפול ח' על ט' יעלו ע"ב והוא חצי קמ"ד שהוא מרובע המורה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועלה בחשבון מחצית אחד שוה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תכפול ב' על ג' יהיה כמו כן מחצית המורה שהוא י"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If you do it with 2 denominators it is easier and there is no need for the square of the denominator, you only look at the product that resulted by multiplying one denominator by the other, consider it as the square, and divide by it. | ואם עשית זה מב' מורים יהיה הדבר יותר קל ואין צורך למרובע המורה רק הסתכל לעולם אל הנכפל העולה מכפל המורה האחד על האחר וחשבהו כמו המרובע ועליו תחלק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון לקחנו המורה האחד ג' בעבור כי אמר שלישיות והמורה האחר ד' בעבור כי אמר רביעיות נכפול המורה האחד שהוא ג' על המורה האחר שהוא ד' ועלו י"ב והוא המבוקש כי העולה נקח ערכו אליו ונקח בעבור הב' שלישיות שנים כי מג' לקחנוהו ומג' רביעיות שלשה כי מד' לקחנוהו ונכפול ב' על ג' עלו ו' והוא חצי מספר הנכפל מהמורים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שאלה כמה ד' שביעיות כפולים על ז' תשיעיות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נבקש מורה אחד לשניהם והנו ס"ג בכפל ז' על ט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וד' שביעיותיו הם ל"ו כי השביעית ט' וז' תשיעיות מ"ט כי התשיעית ז' ונכפול ל"ו על מ"ט עלו אלף ותשס"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומרובע המורה ג' אלפים וט' מאות וס"ט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר חלקנו חשבוננו הראשון על ס"ג עלו כ"ח שהם ד' על ז' והם מן ס"ג ד' תשיעיות שלמות או אם תרצה לומר שהם ג' שביעיות ותשיעית שביעית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם לקחנו בב' מורים יהיה הנכפל ס"ג והעולה בידנו בכפל כ"ח והנה הדבר שוה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If there are fractions of three types: | ואם היו שברים מג' מינים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כמו ב' שלישיות וה' ששיות וד' שביעיות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
קח להם מורה אחד והוא שנכפול ג' על ו' עלו י"ח עוד נכפול י"ח על ז' עלה קכ"ו והוא המורה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה ב' שלישיות קכ"ו פ"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וה' ששיות ק"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וד' שביעיות ע"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפלנו פ"ד על ק"ה והמחובר על ע"ב והעולה מהם בחלוק הוא חלק ממרובע קכ"ו והחלוק יהיה מ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If we take 3 denominators for them, since they are three types, you do not need the square of the denominator, but take the denominator and divide the product of the numbers by it. | ואם נקח להם ג' מורים מפני היותם ג' מינים לא תצטרך למרובע המורה אבל תקח המורה עליו תחלק הנכפל מהמספרים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון כפלנו ב' על ה' עלו י' כפלנו י' על ד' עלו מ' והוא חלק מקכ"ו שהם ב' שביעיות וב' תשיעיות השביעית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
או תעשה כן כפול ג' על ו' והוא י"ח והוא המורה וכפול ב' על ה' יהיו י' נקח ד' שביעיות מי' יהיו ב' שביעיות וב' תשיעיות השביעית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
או כפול ו' על ז' והיו מ"ב והוא המורה וכפול ה' על ד' יהיו כ' נקח ב' שלישיות מכ' יהיו ב' שביעיות וב' תשיעיות השביעית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
או כפול ג' על ז' עלו כ"א והוא המורה ואח"כ כפלנו ב' על ד' עלו ח' לקחנו ה' ששיות והעולה הוא חלק מכ"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If we have integers together with a number that contains no integer but only fractions: | ואם היה לנו שלמים עם מספר שאין שם שלמים כי אם שברים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| We take the fractions from the denominator and give the whole denominator for each integer according to the number of the whole denominator and finally divide by the denominator. | נקח השברים מהמורה ולכל שלם נתן לו מורה שלם כמספר המורה השלם ונחלק באחרונה על המורה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון רצינו לכפול ד' שלמים על ג' חמישיות אחד
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והמורה ה' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ובעבור שיש לנו ד' שלמים נקח להם כ' ונכפול ד' על ג' ונחלק בה' יעלו ב' שלמים וב' חמישיות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If we want to multiply integers and fractions by integers and fractions that are of the same kind. | ואם רצינו לכפול שלמים ושברים על שלמים ושברים שהם ממין אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| We first multiply the integers by the integers, then the fractions by the integers of the one number, likewise the integers of the other number by the fractions of the one number and then the fractions by the fractions. | נכפול בתחלה השלמים על השלמים ואח"כ הנשברים על השלמים של חשבון האחד גם השלמים של חשבון האחר על הנשברים של החשבון האחד ואח"כ הנשברים על הנשברים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Or we convert all into fractions and multiply these by those and divide the product by the square of the denominator. | או נשיב הכל נשברים ונכפול אלה על אלה והעולה נחלקנו על מרובע המורה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון רצינו לכפול ד' שלמים וב' חמישיות על ה' שלמים וג' חמישיות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נכפול תחלה השלמים על השלמים עלו כ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אח"כ נכפול ד' על ג' יהיו י"ב חמישיות שברים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
גם ב' על ה' יהיו י' שברים במיניהם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה כ"ב שברים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואח"כ נכפול השברים על השברים ב' על ג' יעלו ו' והם שברי שברים במעלה השלישית השלמים נחלקם על ה' שהוא המורה עלה שבר אחד ונשאר לנו במעלה השלישית אחד שהוא שבר השבר והשבר שעלה בידנו נחברנו אל כ"ב שהיה לנו הנה כ"ג נחלקם על ה' עלו ד' שלמים ונשארו ג' והנה השלמים כ"ד והשברים ג' שהם ג' חמישיות שהם ט"ו מכ"ה שהוא המרובע ושבר השבר שהוא חומש החומש שהוא והם י"ו מכ"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והדרך האחרת | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
לקחנו לד' השלמים כ' הוספנו עליו ב' שהם השברים עלו כ"ב חומשין והוא החשבון האחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
גם השני על הדרך הזאת כ"ח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נכפול זה על זה ונחלק העולה על המרובע שהוא כ"ה יעלו כ"ד וישארו י"ו שלא יתחלקו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון אחר רצינו לכפול ג' שלמים וד' חומשין על ב' שלמים וג' חומשין | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נכפול תחלה שלמים על שלמים והם ו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואח"כ נכפול שלמים על שברים האלכסונין | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ג' על ג' והם ט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וב' על ד' והם ח' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ובין הכל י"ז שברים ממעלה העליונה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונכפול שברים על שברים ד' על ג' והם י"ב שברי שברים מהמעלה השנית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נחלקם על ה' עלו בידנו ב' שברים והנשאר ב' שברי שברים שלא עלה בחלוק נחבר מה שעלה בחלוק עם השברים שהוא י"ז והם י"ט נחלק על ה' עלו ג' שלמים נחברם אל השלמים שהיו ו' והם ט' נשארו ד' שהם כ' שברי שברים ועם שנים שהיו לנו הם כ"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה סך הכל ט' שלמים וכ"ב שברים מכ"ה השלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Example. We want to multiply integers and fractions by integers and fractions where the fractions are not of the same kind. | דמיון רצינו לכפול שלמים ושברים על שלמים ושברים שאין השברים ממין אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה על הדרך הזה האחד ג' שלמים וד' חמישיות והשני ו' שלמים וז' שמיניות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Multiply the units by the units that are in the first number. | כפול האחדים על האחדים שהם במספר הראשון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Multiply also the fractions by the fractions according to the rule, because the product of the fractions by the fractions is parts of the denominator. | גם כפול השברים על השברים כמשפט כי כפל השברים על השברים הם חלקי המורה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| But, when multiplying the integers by the fractions, we have to remember that their parts are not the same. | רק יש לנו לשמור כשנכפול השלמים על השברים כי אין מחלקותם שוה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה נכפול שלמים על שלמים ג' על ו' עלו י"ח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונכפול עוד אלו ג' על שברי החשבון שהם ז' יעלו כ"א נחלקם על ח' כי שמיניות הם יעלו ב' שלמים והנה ב' שלמים ונשארו ה' שמיניות ידענו כי המורה הוא מ' כי תכפול ה' על ח' ואלה הה' שמיניות כ"ה חלקים כי תכפול ה' על חמישיות שהם ה' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונשוב לכפול הו' על ד' חמישיות יעלו כ"ד נחלקם על ה' יעלו ד' שלמים והנה יהיו השלמים כ"ד וישארו ד' חמישיות והם ל"ב חלקים כשתכפול ד' על ח' נחבר אליהם הכ"ה חלקים שהיו לנו יעלו נ"ז חלקים נעשה מהם אחד שלם מארבעים ויהיו כ"ה שלמים ונשארו י"ז אח"כ נכפול ד' על ז' יעלו כ"ח חלקים נחבר אליהם י"ז יהיו מ"ה והנה נתן אחד שלם ממ' ויהיו השלמים כ"ו והנשאר ה' חלקים שהם שמינית אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The method of the arithmeticians is that they look for one common denominator for fractions that are not of one kind and the number of the denominator is one integer. | ודרך חכמי החשבון שיבקשו לשברים שאינם ממין אחד מורה אחד כולל שניהם ומספר המורה הוא אחד שלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ובעבור כי השברים הם חמישיות ושמיניות יהיה המורה ארבעים והנה נקח לחשבון שהוא ג' ק"כ ולד' חמישיות ל"ב הנה המספר קנ"ב וזה צורת האותיות ונקח לו' השלמים ר"מ ונחבר אליהם ל"ה שהם ז' שמיניות יהיה המספר השני רע"ה והנה נכפול זה על זה והנה עלה מ"א אלף וח' מאות וז' הצורה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקנום על אלף ושש מאות שהוא מרובע המורה עלו כ"ו שלמים נשארו ר' שלא עלו בחשבון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ובקש מהו ר' מן המרובע שהוא אלף ושש מאות והנה הוא שמיניתו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| We can know the remainder in another way: | ונוכל לדעת זה הנשאר בדרך אחרת | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| We always divide the remainder of the square by the denominator itself, so the quotient is parts of it. | שנחלק לעולם הנשאר מהמרובע על המורה בעצמו והעולה הם חלקים ממנו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה נחלק ר' על מ' עלו ה' שהוא שמיניתו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Another method with 2 denominators: | דרך אחרת מב' מורים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה נשים החשבון האחד שהוא ג' ט"ו ונחבר אליהם ד' יהיה החשבון הראשון י"ט נקח לששה מ"ח נחבר אליהם ז' יעלו נ"ה והוא החשבון השני נכפול זה על זה יעלו אלף ומ"ה נחלק אותו על הנכפל שהוא כפל ה' על ח' והוא מ' ויעלו כ"ו שלמים נשארו ה' שהוא שמינית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Now, we should speak of integers and fractions that man cannot express. | ועתה יש לנו לדבר על שלמים ונשברים שלא יוכל האדם לבטא בהם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If one of the fractions is one that can be expressed and the other is one that cannot be expressed, then proceed as follows: | והנה אם היה אחד מהשברים שיוכל לבטא בהם והאחרים שלא יוכל לבטא בהם יעשה ככה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון כמה שלש שביעיות אחד על ה' חלקים מי"א כי הוא השלם ולא יוכל אדם לבטא בו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה נכפול השברים על השברים והנה יהיה המורה ע"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה יש לנו להשמר כי השברים יהיו להפך כי כל אחד מהז' יהיו י"א וכל אחד מהי"א יהיו ז' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה נקח בעבור ג' שביעיות ל"ג ובעבור ה' חלקים מי"א נקח ל"ה והנה נכפול אלה על אלה עלו אלף קנ"ה נחלקם על ע"ז עד שנדע כמה הם השברים העולים מזה הכפל אל ערך ע"ז שהוא השלם והם ט"ו והנה מן ע"ז פחות מעט מחמישית אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועל דרך דקדוק יפה נחלק אלה ט"ו על י"א שהוא השביעית יעלה שביעית אחד שלם וד' חלקים מי"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם נחלק על ז' יעלו ב' חלקים מי"א ושביעית חלק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון אחר לב' שברים שלא יוכל האדם לבטא בהם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נשים החשבון האחד י"ג והשני י"ט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה בקשנו לכפול ט' חלקים מי"ג על י"ז חלקים מי"ט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נבקש בתחלה המורה בזה הדרך שנכפול י"ג על י"ט ועלה המספר רמ"ז והוא המורה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אח"כ כפלנו ט' בי"ט ועלה קע"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכפלנו י"ז בי"ג ועלה רכ"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה כפלנו זה על זה והיה העולה ל"ז אלפים ותשצ"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקנום על רמ"ז והיה קנ"ג והנה העולה מכפלנו ט' בי"ז הוא ג"כ קנ"ג וערך זה אל רמ"ז כערך המספר הראשון אל המרובע רמ"ז והוא המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תרצה לדקדקנו חלק קנ"ג על י"ט ויהיו ח' חלקי' מי"ג וחלק אחד מי"ט בי"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
או אם תרצה חלקהו על י"ג ויהיו י"א חלקי' מי"ט וי' חלקים מי"ג בי"ט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If you have integers and fractions that are like this, proceed in the way that I have shown you when you have integers and fractions that you can express. | ואם היו לך שלמים עם שברים שהם בדרך זה עשה כדרך שהראיתיך כשיהיו לך שלמים עם שברים שתוכל לבטא בהם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| I mentioned this method because it is very necessary for most problems and for the matters of the square numbers to know their roots, when they are fractions that man cannot express, as I will explain in chapter seven. | והזכרתי זה הדרך כי צורך גדול יש אליו ברובי השאלות ובדברי המרובעים לדעת שרשיהם כשהם נשברים ולא יוכל האדם לבטא בהם כמו שאפרש בשער השביעי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| I shall tell you a general method for a fraction of fractions of fractions and give you one example that is enough for you, because it is not needed for proportions, neither for roots, nor for problems. | ואומר לך דרך כוללת לשבר שברי הנשברים ואתן דמיון אחד ויספיק לך כי אין צורך לדבר הזה בערכים ולא בשרשים ולא בשאלות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון כפלנו ב' שלישיות רביעית חמישית על שש (ד') שביעיות שמינית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה השברים רבים אך אלמד לך דרך קצרה איך תעשה [47]
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דע כי מאחר שיש לך ששיות ושמינית אין צורך לשלישית ורביעית והנה נבקש חשבון שיש לו חמישית וששית ושביעית ושמינית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נכפול ה' על ו' יעלו ל' גם ל' על ז' יהיו ר"י גם ר"י על ח' יעלו אלף ו' מאות ופ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה נבקש המספר הראשון והנה חמישית המורה של"ו ורביעיתו פ"ד וב' שלישיותיו נ"ו וזהו החשבון האחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכבר ידענו כי השמינית ר"י ושביעיתו ל' ובעבור שהם ד' שביעיות יהיה המספר השני ק"כ | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נכפול זה על זה יהיה המספר י' (ו') אלפים ופ' (תש"כ) חלקנו זה על אלף ו' מאות ופ' עלו ו' (ד') ואלה הו' (ד') הם חמישית שמינית (חצי ששית) השביעית שהשביעית ר"מ והשמינית ל' והחמישית ו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Now we talk again about the division of the fractions. Do as I have shown you, by turning the fractions into whole units. | ועתה נשוב לדבר על חלוק השברים עשה כדרך שהראיתיך שתשיב הנשברים אחדים שלמי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If there are integers with the fractions, proceed according to the instructions. | ואם היו שלמים עם השברי' עשה כמשפט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון בקשנו לחלק ג' שלמים ושתי חמישיות על ב' שלמי' וד' שביעיות אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה המורה ל"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והג' שלמים ק"ה וב' חמישיות י"ד והנה החשבון קי"ט ונשיב הב' השלמים האחדים ע' והד' שביעיות כ' הנה צ' חלקנו קי"ט עליו עלה אחד שלם ונשארו כ"ט שהם שתי תשיעיות ועשירית אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון לנשברים לבדם חלק ז' תשיעיות על ב' שביעיות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה המורה ס"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ושבע תשיעיותיו מ"ט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וב' שביעיותיו י"ח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקנו זה על זה עלו ב' וו' תשיעיות וחצי תשיעית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| You proceed in this way, because there is no great need to divide fractions. | ועל זה הדרך תעשה כי אין צורך גדול לחלק הנשברים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| We talk again about their addition. | ונשוב לדבר על חבורם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חברנו ב' חמישיות אחד על ה' שביעיות אחד כמה העולה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה המורה ל"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וב' חמישיותיו י"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וה' שביעיותיו כ"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נחברם והנה ל"ט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נקח בעבור ל"ה אחד שלם ונשארו ד' שהם ד' חמישיות מהשביעית שהם אחד מל"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| How Much Problem - Money | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שאלה חברנו אל ממון תשיעיתו ועשירתו והיו נ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נשים המורה צ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וידוע כי תשיעיתו ועשיריתו י"ט נוסיפם על צ' יהיו ק"ט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה גם נשיב הנ' מערך התשיעית יהיו ד' מאות ונ' נשיב הד' מאות ונ' מערך העשירית יהיו ד' אלפים ות"ק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נחלק זה על ק"ט ויעלו מ"א שלמים גם ל"א חלקים מזה שחלקנו עליו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה נבחן אם זה אמת | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ידענו כי עשירית מ' ד' שלמים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונקח לאחד שנשאר ק"ט נחבר אליו ל"א שהם יתרים על השלמים הנה ק"מ ועשיריתם י"ד ואלה הם חלקי העשירית הנוספים על השלמים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונשוב לקחת התשיעית והנה מל"ו ד' שלמים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונשארו ה' שלמים נשים כל אחד ק"ט יהיו תקמ"ה נחבר אליהם ל"א היתרים על השלמים יהיו תקע"ו ותשיעיתם ס"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נחבר אליהם י"ד שעלו מן העשירית יהיו ע"ח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
גם נחבר אליהם ל"א היתרים יהיו ק"ט והנה אחד שלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה הכל נ' שלמים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שאלה אחרת לקחנו חמישית ממון גם שביעיתו ותשיעיתו כמה הוא מערך הממון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תוכל להוציא שאלה זו על שני דרכים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
האחד שהתשיעית פחותה משאר השברים האחרים הנה נחשוב כי הם ג' תשיעיות והיתרון שיש בין החמישית והתשיעית ד' [48] והיתרון שיש בין החמישית והשביעית ב' נכפול ב' על ד' יעלו ח' נעשה מן הז' תשיעית אחת יהיו ד' תשיעיות שלמות ונשאר אחד והיתרון בין השביעית (?) והתשיעית (?) ב' והנה הממון הוא ד' תשיעיות וג' חלקים שהם ג' חמישיות שביעית תשיעית
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והדרך האחרת שנבקש מורה שנכפול ה' על ז' יהיו ל"ה גם נכפול זה על ט' יעלו שט"ו והוא המורה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואח"כ נחבר חמישית זה המורה ושביעיתו ותשיעיתו יהיו קמ"ג נחלקם על ל"ה והנה הם ד' תשיעיות ונשארו ג' חלקים מל"ה כי ל"ה הוא התשיעית וה' הוא שביעית התשיעית ולכן ג' חלקים הם ג' חמישיות שביעית התשיעית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שאלה אחרת ממון הוספנו עליו מחציתו ושלישיתו וחמישיתו וששיתו ובין הכל היו מ' כמה היה הממון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ידענו כי החצי והשלישית והששית הוא אחד שלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונחשוב כי היה לו אחד והנה שנים ויש לנו תוספת החמישית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה יש לנו לחלק מ' על ב' וחמישית והעולה הוא הממון והנה נקח לכל אחד מהשלמים ה' ונשים עמהם א' שהוא החמישית יהיו י"א גם נכפול מ' על ה' עד שיהיו דרך אחד יהיו ר' נחלקם על י"א יעלו י"ח שלמים ועוד ב' חלקים מי"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נבחן זה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נקח חציו שהוא ט' שלמים וחלק אחד מי"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה יהיה לנו כ"ז שלמים וג' חלקים מי"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והשלישית ו' שלמים וב' שלישיות אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה יהיו לנו ל"ג וג' חלקים וב' שלישיות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והחמישית מט"ו נקח ג' שלמים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
היו לנו ל"ו שלמים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונשאר לנו לקחת חמישית ג' שלמים והנה נקח לכל אחד י"א ונחבר אליהם הב' חלקים היתרים יהיו ל"ה וחמישיתם ז' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נחבר אליהם הג' חלקים שהיו לנו וב' שלישיות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונקח ששית י"ח יהיו ג' שלמים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נחברם אל ל"ו יהיו ל"ט שלמים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ושתי ששיות הם שליש אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נחברנו אל י' חלקים וב' שלישיות חלק שהיו לנו יהיו י"א חלקים והוא אחד שלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והכל מ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| How Many Problem - Group of People | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| A man passed by a group of people. He said to them: hello one hundred people. They answered him: we are not one hundred people, but all of us, and other like us, and half of us, and a quarter of us together with you would be one hundred.
|
שאלה אדם עבר על אנשים אמר להם שלום עליכם מאה איש ענו לו אין אנחנו מאה רק אנחנו ואחרים כמונו ומחציתנו ורביעיתנו ועמך נהיה מאה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה נקח למספרם אחד ואחד כמוהו הנה שנים וחצינו חצי אחד הנה שנים וחצי נוסיף רביעיתנו הנה יהיו שנים וג' רביעית ובעבור שיש לנו רביעיות נקח לכל אחד שלם ד' יהיו ח' ונחבר אליהם הג' רביעים יהיו י"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ובעבור שאמרו כי עמו יהיו מאה יהיה מספרם עם התוספת (ת)צ"ט נשיבם מדרך הד' יהיו שצ"ו נחלקם על י"א יהיו ל"ו וככה מספרם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Buy and Sell Problem | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שאלה אדם קנה בק' זהובים ק' ליט' ואח"כ מכר הנ' ליט' ורביע בזהוב והנ' האחרים מכר ליט' פחות רביע בזהוב נבקש לדעת אם הרויח או הפסיד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נשיב הנ' ראשונים ר' כי רביעיים הם נחלקם על ה' כי ליט' ורבי' ליט' מכר בזהוב ויהיו מ' זהובים גם נכפול הנ' האחרי' על ד' יהיו ר' נחלקם על ג' כי ג' רביעיי' מכר בזהוב והנה יהיו ס"ו זהובים ושתי שלישיות זהוב נחבר אליהם המ' יהיה הריוח ו' זהובים ושתי שלישיות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שאלה אחרת אדם קנה ג' חמישיות ליט' בפשוט ומכר ד' שביעיות ליט' בפשוט והרויח פשוט כמה היה ממונו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
בקש המורה והוא ל"ה שהוא כפל ה' על ז' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה ג' חמישיותיו כ"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וד' שביעיותיו כ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והממון היה כ' והליט' י"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שאלה אדם קנה ד' שביעיות ליט' בפשוט ומכר אותם ה' תשיעיות ליט' בפשוט והרויח פשוט כמה היה הממון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ידוע כי ד' שביעיות אחד יותר מה' תשיעיות אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה המורה ס"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וה' תשיעיותיו ל"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וד' שביעיותיו ל"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותוכל לבחון זה כי אחר שקנה ד' שביעיות ליט' בפשוט וממונו ל"ה הנה יש לו כ' ליט' עשה מהם תשיעיות יהיו ק"פ חלק זה המספר על ה' כי ה' תשיעיות מכר בפשוט יעלה בידך ל"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואלו אמר כי הרויח ב' פשוטים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפלם על ל"ה יהיו ע' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם אמר ג' פשוטים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
יכפול על ל"ה ג' פעמים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| And so on to the end of all numbers | וככה עד סוף החשבון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שאלה אדם קנה ט' חלקים מי"ז חלקי ליט' בפשוט ומכרם י' חלקים מי"ט חלקי ליט' בפשוט והרויח פשוט כמה היה הממון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
בקש המורה והוא שכ"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ידוע כי ט' חלקים מי"ז הם קע"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וי' חלקים מי"ט הם ק"ע וכך היה הממון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והליט' צ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The following reason of subtraction is easy: | וזה דבר המגרעת קל הוא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| For you set one denominator for two fractions, then consider the denominator as one integer. | כי תשים מורה אחד לב' שברים והמורה תשימנו אחד שלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון רצינו לגרוע ד' תשיעיות מה' שביעיות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
המורה ס"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וה' שביעיותיו מ"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וד' תשיעיותיו כ"ח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חסרנו כ"ח ממ"ה ישארו י"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Now, we shall discuss the method of astrology again | עתה נשוב לדבר על דרך חכמת המזלות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Make sure you understand their ways; because Ptolemy and his colleagues found the way to the roots of square numbers only according to them. | ושים לבך להבין דרכיהם כי תלמי וחביריו לא מצאו דרך שרשי המרובעים אלא על פיהם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Know that man can divide every circle and what is non-circular into as many parts as he wants, depending on his need and desire. | ודע כי כל עגול ומה שאיננו עגול יכול האדם לחלקו על כמה חלקים שירצה כפי חפצו וצרכו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The arithmeticians found no smaller number that has many parts that are integers, only 12, because it has a half, a third, a quarter, a sixth and a half of a sixth. | והנה חכמי החשבון לא מצאו חשבון קטן שיש לו חלקים רבים שהם אחדים שלמים רק י"ב כי יש לו חצי ושלישית ורביעית וששית וחצי ששית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| This is because there is no number smaller than it, whose factors add up to more than itself, only it alone, because its factors exceeds by one-third over itself. | והיה כן בעבור שאין חשבון פחות ממנו שיהיו חלקיו רבים ממנו רק הוא לבדו כי חלקיו יוסיפו עליו שלישית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 120 is its analogous number in the tens; therefore, its factors are twice the number, no more nor less. | וק"כ דומה לו בעשרות על כן חלקיו כפל המספר בלי תוספת ומגרעת | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| That is why the astrologers divided the sphere into 12 parts. | ע"כ חלקו חכמי המזלות הגלגל לי"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| They named each sign after the shape of the constellation of the highest stars, which are close to the line of the celestial circle. | וקראו כל מזל בשם צורת הכוכבים העליונים שהם קרובים לקו גלגל המזלות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Also because they found that in the solar year the moon reappears 12 times. | ועוד כי מצאו בשנת השמש שתתחדש הלבנה י"ב פעמים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| They divided the celestial sphere into 360 degrees, because this number is close to the number of the days of the solar year. | וחלקו הגלגל על ש"ס מעלות שזה המספר קרוב למספר ימי שנת החמה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| There is no number smaller than it that has all the parts that man can express except the seventh. | ואין מספר פחות ממנו שכל החלקים שיבטא אדם בהם יש לו חוץ מהשביעית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Therefore, when this number is multiplied by 7, the result is 2520 and this is the number that has all parts. | ע"כ כשיכפול זה החשבון על ז' יעלה אלפַים וה' מאות וכ' וזהו המספר שיש לו כל החלקים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Often the arithmeticians need it. | ופעמים רבות שיצטרכו בעלי החשבון אליו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| As saying: A number, We have added to it all of its parts from half to tenth; what is the ratio of the sum to it? | כאומר חשבון חברנו אליו כל החלקים מחצי עד עשירית מה ערך המספר אליו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| This whole number is considered as one integer. | כי יחשוב זה החשבון הכולל כי הוא אחד שלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| When we divide the celestial sphere into 12 parts, each sign has 30 degrees and there is no number smaller than it that has so many parts as it, because it has a half, a third, a fifth, a sixth and a tenth. | וכאשר חלקנו הגלגל על י"ב עלה לכל מזל ל' מעלות ואין מספר פחות ממנו שיש לו חלקים רבים כמוהו כי יש לו חצי שלישית חמישית ששית ועשירית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Since it does not have a quarter, this number has been doubled, so it become 60. | ובעבור שאין לו רביעית כפלו זה החשבון והיה ס' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Therefore, they divide each degree by it, which is as a sixtieth, and they called them "primes". | על כן חלקו כל מעלה ממנו שהיא כמו אחד על ששים חלקים וקראו אותם חלקים ראשונים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| They divided each prime to 60 and called them "seconds". | וחלקו כל ראשון לששים וקראום שניים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Likewise, they divide each second into 60 and called the result "thirds". | גם ככה עשו על כל שני על ששים והעולה יקראו שלישיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| They proceed so to ten, each [is divided] in sixtieths, and even further if necessary. | וככה יעשו עד עשרה כל אחד לששים ויותר אם יצטרכו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Now, it should be discussed about how they are multiplied one by the other. | ועתה יש לדבר איך יכפלו זה על זה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Always consider the degrees as if they are units of integers. | ולעולם חשוב כי המעלות הם כמו אחדים שלמים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| So, if you multiply degrees by degrees, the product is degrees. The product of degrees by minutes is minutes, and by seconds is seconds. | והנה אם כפלת מעלות על מעלות יהיה הנכפל מעלות וכפל מעלות על ראשונים ראשונים ועל שניים שניים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The rule: the product of degrees by any type is the same type itself. | והכלל כפל מעלות על איזה מין שיהיה יעמוד אותו המין בעצמו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The product of minutes multiplied by minutes is seconds, and the product of minutes by seconds is thirds and in this way for all types. | וכפל ראשונים על ראשונים יהיה העולה שניים וראשונים על שניים יהיה העולה שלישיים ועל זה הדרך כל המינין | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The product of seconds multiplied by seconds is fourths, and by thirds is fifths. | וכפל שניים על שניים יהיה הנכפל רביעיים ועל שלישיים חמישיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The product of thirds by thirds is sixths, and so is the product of thirds by fourths sevenths. | וכפל שלישיים על ששיים ששיים עד שיהיה כפל שלישיים על רביעיים שביעיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Fifths by fifths or thirds by sevenths is tenths. | וחמישיים על חמישיים או שלישיים על שביעיים עשיריים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Now I shall give you two correct methods: | והנה אתן לך שנים דרכים נכונים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The first method by writing: | הדרך האחד במכתב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Set the degrees in the first rank, the minutes in the second rank and so on, all fractions successively. | שתשים המעלות במעלה הראשונה והראשונים במעלה השנית וככה כל השברים זה אחר זה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| This writing is opposite to what we do in the multiplication of integers, because the smallest number is in the first [rank]. | וזה המכתב בהפך מה שאנו עושים בכפל השלמים כי החשבון המעט הוא בראשונה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If you have no degrees, write a zero in the first [rank]; if you have no minutes, write a zero in its place, and so you do it at the place of each fraction, if there is a smaller fraction after it. | ואם אין לך מעלות כתוב גלגל בראשונה ואם אין לך ראשונים כתוב גלגל במקומו וככה תעשה במקום כל שבר אם יש שבר אחריו פחות ממנו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| With this method you can know in which rank of the fractions any fraction will stand. | ובזה הדרך תוכל לדעת באיזו מעלה מן השברים יעמד איזה שבר שתרצה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Proceed as with integers, but you have to make sure that you put a vertical line between all types of fractions. | ועשה כדרך השלמי' רק יש לך להשמר שתשים קו באורך בין מיני השברים כלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If there are 2 digits of whichever type of fractions, such as 22, when you write them down, you have to do like this: set them breadthwise between the two lines according to their rank, as you do it with integers. | ואם היו ב' חשבונים באיזה מין שיהיה מן השברים כגון כ"ב יש לך לעשות כשתכתבם שים אותם ברוחב שבין שני הקוים במעלתם על הדרך שאתה עושה בשלמים כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| We have already drawn lines between the fractions and arrived to thirds for the upper rows and also for the lower rows. | הנה כבר שמנו קוים בין השברים והגענום עד שלישיים ככה הטורים העליונים וככה השפלים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| When we multiply the upper rows by the lower rows, their product is the number that is written in the bottom. | וכאשר כפלנו הטורים העליונים על השפלים עלה מספרם המספר הכתוב למטה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| We divide each rank by 60, then add the quotient to what is in the preceding rank, and write the remainder alone. We do this with each rank until we arrived to the degrees that are as the integers. | חלקנו כל מעלה על ששים וחברנו העולה על ההוה במעלה הראשונה והנשאר כתבנו לבדד וככה עשינו מכל מעלה ומעלה עד שהגענו למעלות שהם כמו השלמים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| So, of sixths remain 33, of the fifths 56, of the fourths 31, of the thirds 24, of the seconds 30, of the minutes 7 and of the degrees also 7. | והנה נשארו מן הששיים ג'ג' ומן החמישיים ו'ה' ומהרביעיים א'ג' ומהשלישיים ד'ב' ומהשניים 0ג' ומהראשונים ז' ומהמעלות גם כן ז' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The second method is by expression: | והדרך השנית במבטא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| You sum the two fractions by adding the ranks of the fractions. | שתחבר השנים השברים ובמחברתם מעלות השברים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| When we do according to the method of the arithmeticians that we convert the upper rows into thirds, the resulting number is: 464643. | וכאשר עשינו על דרך חכמי החשבון שהשיבונו הטורים העליונים אל שלישיים עלה החשבון כך ג' ד' ו' ד' ו' ד' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Likewise, we do it with the lower [rows], the resulting number is: 715451. | ככה עשינו בשפלים עלה החשבון כך א' ה' ד' ה' א' ז' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| We multiply one by the other, the resulting number is: 332429298993; it has 12 rows. | והנה כפלנו זה על זה ועלה המספר כזה ג'ט' ט'ח' ט'ב' ט'ב' ד'ב' ג'ג' והם י"ב טורים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| All these are sixths; we divide them by 60 and the result are fifths: 5540488316, and 35 sixths remain in the first number. | והנה כל אלה ששיים חלקנום על ס' ועלו חמישיים כזה ו' א' ג' ח'ח' ד'0 ד'ה'ה' ונשארו ל"ה ששיים במספר הראשון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| We proceed so successively; the number resulting last and what is left of each rant will be as the number in the first method. | וככה עשינו על זה הסדר והיה המספר שעלה באחרונה גם הנשאר מכל מעלה ומעלה כמספר הדרך הראשונה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| I had to show the method of these parts, which are minutes to thirds, because with this method King Ptolemy calculated the chords of the arcs until reaching to fifths, which are by multiplying tenths. | והוצרכתי להראות דרך אלה החלקים שהם הראשוני' עד שלישיים כי על זה הדרך הוציא תלמי המלך יתרי הקשתות עד שהגיעם אל חמישיים והם בכפל עשיריים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| He also did all of this to extract the roots of the square numbers that do not have a real root truly, but it is only approximated to the truth, because there are no minutes or seconds left in dividing the product, not even thirds, as I will explain in the seventh chapter. | וכל זה עשה להוציא שרשי המרובעים שאין להם שורש נכון באמת רק יוציאוהו קרוב אל האמת כי לא ישארו בחלוק הכפל ראשונים ולא שניים ואפילו שלישיים כאשר אפרש בשער השביעי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Know that in every science there are things that are hidden from the eyes of all the ancient sages and also those that follow them. | ודע כי יש בכל חכמה דברים נעלמו מעיני כל החכמים הקדמונים וגם מכל הבאים אחריהם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Such as: the roots of the numbers that are not square numbers, in arithmetic. | כמו שרשי המספרים שאינם מרובעים בחכמת החשבון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| As well as in geometry, to know the the perimeter from a known diameter. | וככה בחכמת המדות לדעת הקו הסובב מאלכסון ידוע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Archimedes the wise could not approximate it to the truth, but only gave evidences from geometry that the perimeter should be three times the diameter plus an excess of 10 parts of 70 of the first rank, and he brought evidences that they had to be less than one part of the seconds, but did not know how many they are, only proved that the excess had to be more than 10 parts of 70 parts and one-half of the part. | ולא יכול ארישמדס החכם לקרבו אל האמת רק שנתן ראיות מחכמת המדות כי הקו הסובב ראוי להיותו שלשה מהאלכסון ותוספת י' חלקים מע' במעלה אחת והביא ראיות שיפחתו מחלק אחד מאלו החלקים שניים ולא ידע כמה הם רק הביא ראיה כי התוספת ראויה להיות יותר י' חלקים מע' חלקים וחצי חלק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| King Ptolemy took the middle path and therefore said that the excess is 8 parts of 63, and 30 seconds. We shall discuss it at the end of the seventh chapter. | ותלמי המלך תפס הדרך האמצעית על כן אמר כי התוספת היא ח' חלקים מס"ג גם ל' שניים ובסוף השער השביעי נדבר על זה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| So also in astrology sages found, by experiment on herbs, stones and the joints of the human body that are true, but none of them knows why this is so, only the sublime God alone. | וככה בחכמת התולדת מצאו חכמים בדרך נסיון בעשבים ובאבנים ובבתי אברי הגוף האדם והם אמת ואין אחד מהם יודע למה היה ככה רק השם ית' הנשגב לבדו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Chapter Six - Proportions |
השער הו' הוא שער הערך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The types of the proportions are according to three ways: | מיני הערכי' על ג' דרכים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
האחד ערכי החשבון והם על הסדר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כמו א' ב' ג' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כי לא יתכן להיות הערך פחות מג' מספרים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
או ב' ד' ו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
או ג' ו' ט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והטעם כי הערך בכלם שוה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
פי' כי כיתרון ד' על ב' כן יתרון ו' על ד' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והערך השני ערכי המדות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כמו ד' ו' ט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כי ערך ד' אל ו' כמו ערך ו' אל ט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כן כפל הקטן אל הגדול ככפל התיכון על עצמו שהוא מרובעו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ודע כי אלה הג' מספרים הם כמו ד' כי האמצעי יחשב כאלו הוא מספר אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
על כן כל ד' מספרי' שערך הראשון אל השני כערך השלישי אל הרביעי אם תחבר מרובעי ארבעתן ותדע כמה יעלה ותחבר הראשון עם הרביעי ותקח מרובע המחובר ותוסיף עליו מרובע היתרון שיש בין השני ובין השלישי יהיה שוה כעולה בראשונה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Likewise, if you sum the second and third, take the square of the sum and add to it the square of the difference between the first and fourth, the result is the same as the first [result]. | וככה אם תחבר השני והשלישי ותקח מרובע המחובר ותוסיף עליו מרובע היתרון שיש בין הראשון ובין הרביעי ימצא העולה שוה לראשון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון ערך ד' על ו' כערך ח' אל י"ב [note 83]
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה מרובעיהם ר"ס והמחובר מן הראשון והרביעי י"ו ומרובעו רנ"ו והיתרון בין ו' וח' הוא ב' ומרובעו ד' והנה המספר שוה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וככה המחובר מן השני והשלישי י"ד ומרובעו קצ"ו והיתרון בין הראשון והרביעי ח' ומרובעו ס"ד והנה המספר שוה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ודע כי רוב חכמת המזלות ותקוני מקום המשרתים תלויים בחכמת ערכי המדות וככה רובי דיני השאלות בחשבון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והדרך השלישי ערכי חכמת הנגינות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והיא חכמה מפוארת מאד כי ערכיה מורכבים מערכי החשבון וערכי המדות [note 84]
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כי לעולם יהיה הערך שבין הראשון לתיכון אל ערך שיהיה בין התיכון [49]לאחרון כערך המספר הראשון למספר האחרון [note 85]
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון ב' ג' ו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ידענו כי הערך שבין ב' וג' אחד והערך שבין ג' ובין ו' שלשה וככה ערך ב' אל ו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון אחר ג' ד' ו' או אם תרצה כ' ל' ס' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה הערך שבין ג' וד' אחד והערך שבין ד' וו' שנים והנה הוא כפלו וככה ו' אל ג' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If you know two numbers, you can extract the third. | והנה אם ידעת השנים מספרי' תוכל להוציא השלישי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כי אם ידעת הראשון והשני ולא תדע השלישי כפול הראשון על השני והעולה חלקנו על הראשון אחר שתגרע ממנו פי' מהראשון היתרון שיש בינו ובין השני[note 86]
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון הראשון כפלנו ב' על ג' וחלקנו העולה על הראשון אחר שגרענו ממנו היתרון שבין הראשון והשני והוא אחד והנה היו ו' והוא השלישי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ובדמיון השני כפל ג' על ד' היו י"ב והיתרון בין ג' וד' אחד גרענוהו מג' שהוא הראשון ונשארו ב' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונאמ' כי אם ידענו השני והשלישי ולא ידענו הראשון נכפול אלה הידועי' שהם השני והשלישי ונחלק העולה על המחובר מהמספר השלישי עם היתרון שיש בין השני והשלישי והעולה הוא הראשון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה בדמיון הראשון כפלנו ג' על ו' והעולה י"ח והיתרון שבין השני והשלישי שלשה חברנום אל ו' והיו ט' חלקנו י"ח עליו עלו ב' והוא הראשון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ובדמיון השני כפלנו ד' על ו' והיו כ"ד חלקנום על ח' שהוא כמספר החשבון השלישי עם היתרון שיש בין השני והשלישי עלו ג' והוא הראשון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם לא ידענו האמצעי ונבקש לדעתו נכפול הראשון על השלישי ונחלק העולה על המחובר מן השנים מספרים והעולה בחלוק נכפלנו והוא המספר האמצעי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון הראשון כפלנו ב' על ו' עלו י"ב חלקנו על ח' שהוא המחובר משניהם עלה א' וחצי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ובדמיון האחר כפלנו ג' על ו' עלו י"ח חלקנו על ט' שהוא המחובר משניהם עלו ב' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וככה בערכי המדות אם לא ידענו האחד מן הד' נוכל להוציאו מן הג' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולעולם נשים שתי הקצוות שהם הראשון והרביעי חברים והשנים האמצעיי' שהם השני והשלישי חברים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה אם לא ידענו אחד מן הקצוות איזה מהם שיהיה נכפול האמצעי על חבירו והעולה נחלקנו על אחד מהקצוות שהוא נודע והעולה הוא המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם לא ידענו אחד מהאמצעיים נכפול אחד מהקצוות על חברו ונחלק העולה על האמצעי הנודע אז ימצא המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| ועל זה הדרך תעשה בכל השאלות ויש לך להשמר באיזה מקום תשים הגלגל | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Word Problems |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Now I shall write a lot of problem for you to practice | ועתה אכתוב לך שאלות רבות כדי להרגילך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| How Much Problem - Money | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Question: a fifth, a sixth, and a seventh of an amount of money make 10, how much is the money?
|
שאלה ממון חברנו חמישיתו וששיתו ושביעיתו והיו עשרה כמה הממון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
בקשנו המורה שכפלנו ה' על ו' עלו ל' גם[50] זה על ז' והיו ר"י והוא המורה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וחמישיתו מ"ב וששיתו ל"ה ושביעיתו ל' והנה שלשתם ק"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה ערך הממון שהוא עשרה המחובר מהם אל כל הממון שאינו נודע כערך ק"ז אל ר"י פי' שהוא המורה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונעשה הדמיון כך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפלנו הקצוות שהם י' על ר"י והיו אלפי' וק' חלקנום על האמצעי שהוא ק"ז עלו י"ט שלמים ונשארו ס"ז חלקי' מן ק"ז והוא כל הממון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואלו היינו עושים להפך היה הדבר שוה כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונבחן זה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כי ג' שלמים חמישית ט"ו ונשארו ד' שלמי' שלא לקחנו חמישיתם נעשה מן כל אחד ק"ז ונחבר אל המחובר ס"ז יהיה הכל תצ"ה וחמישיתם צ"ט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וששית י"ח ג' ונקח לא' הנשאר ק"ז נחבר אליו ס"ז יהיו קע"ד וששיתו כ"ט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
גם השביעית שנים שלמי' נשארו ה' שאין להם שביעית נקח לכל אחד ק"ז יהיו תקל"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נחבר החלקים יהיו שנים שלמים נחברם אל השלמים והיו י' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Another example: We took its seventh and its ninth; they are 7.
|
דמיון אחר לקחנו שביעיתו ותשיעיתו והיו ז' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
המורה ס"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והשביעית והתשיעית י"ו וככה הצורה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה החשבון כ"ז שלמים וט' חלקי' מי"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| First from Last Problem - Money | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If one reversed the saying and says: We have subtracted from an amount of money its seventh and its ninth and 7 remain.
|
ואם הפך הדבר ואמר חסרנו מהממון שביעיתו ותשיעיתו נשארו ז' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
גם אנו נעשה המורה להפך כי המורה הוא אחד רק נחסר י"ו שהוא השביעית והתשיעית מהמורה ונשארו מ"ז ונכתבהו ככה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפלנו ז' על ס"ג והיו תמ"א חלקנו על מ"ז עלו ט' וי"ח חלקי' ממ"ז ועתה הוא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ושביעיתם כדרך שהראיתיך עלה אחד שלם גם י"ו חלקי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
לקחנו תשיעיתו והוא אחד שלם וב' חלקי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונשארו ז' שלמים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Partnership Problem - For the Same Time | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Question: 4 people - one of them have 11 dinar, the second have 13 dinar, the third have 15 dinar, and the fourth have 17 dinar. They earned 19 dinar. How much does each get? | שאלה ד' אנשים יש לאחד מהם י"א דינ' ולשני י"ג דינ' ולשלישי ט"ו דינ' ולרביעי י"ז דינ' והרויחו י"ט דינ' כמה יקח כל אחד ואחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נבחר ראשי כל ממונם והיו נ"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכערך כל אחד אל נ"ו ככה יקח מי"ט ונעשה כך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נכפול י"א על י"ט יעלו ר"ט נחלק על נ"ו עלו ג' שלמי' ונשארו מ"א חלקי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עשינו כן בי"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עלו רמ"ז חלקנום על נ"ו עלו ד' שלמי' וכ"ג חלקי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עשינו כך בט"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועלו רפ"ה חלקנום על נ"ו עלו ה' שלמי' וה' חלקי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עשינו כך בי"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עלו שכ"ג חלקנום על נ"ו עלו ה' שלמים ומ"ג חלקים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חברנו השלמי' ואלה החלקי' ועלו י"ט שלמי' כי אלה החלקי' חלקי נ"ו הם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דרך אחרת השיבונו הריוח כלו פשוטים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שכפלנו הי"ט דינ' בי"ב עלו רכ"ח חלקנום על נ"ו עלו ד' פשוטי' גם ד' חלקי' מנ"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה כפלנו י"א על ד' עלו מ"ד פשוטי' שהם ג' דינ' ח' פשוטי' ומ"ד חלקי פשוט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
גם כפלנו י"ג על הד' והיו נ"ב שהם ד' דינ' וד' פשוטי' ונ"ב חלקי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
גם כפלנו ט"ו על ד' והיו ס' פשוטי' שהם ה' דינ' וס' חלקי' חברנו פשוט מנ"ו ושארו ד' חלקים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
גם כפלנו י"ז על הד' והיו ס"ח פשו' שהם ה' דינ' וח' פשו' וס"ח חלקי' נחבר מהם פשוט ישארו י"ב חלקים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נחבר החלקים כלם יהיו ג' פשו' ונחבר הפשוטי' יהיו ב' דינ' נחברם אל החלק הגדול יהיו בין הכל י"ט דינ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Purchase Problem – Moneychanger | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Question: the moneychanger has three [kinds of] coins. One zahuv is worth three dinar of the first [kind of] coins; or four of the second [kind]; or six of the third [kind]. A man came and asked the moneychanger to give him from the three [kinds of] coins for one zahuv equally, so that the amount of the expensive will be equal to the amount of the inexpensive.
|
שאלה יש אצל המחליף שלשה המטבעים שוה הזהוב ממטבע האחד ג' דינ' וממטבע השני ד' דינ' וממטבע השלישי' ו' דינ' ובא אדם אחד ובקש למחליף שיתן לו מג' המטבעי' בזהוב ויהיה המספר בשוה מן היקרים כמו משאינם יקרים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
בקש חשבון שיש לו שלישית ורביעית וששית והוא י"ב והוא המורה וכל החלקים הנזכרים הם ט' והוא דינר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|
ונבקש מה ערך י"ב אל ט' והנה הוא כמוהו ושלישיתו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|
והנה נוסיף על י"ב פשו' שהוא דינר ד' פשו' שהוא שלישיתו ועלו י"ו פשו' וככה לקח מכל מטבע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולבחון דבר זה קל הוא כי הערכי' נמצאים במהרה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| והנה נשים המטבע של שלשה עקר | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וידענו כי הי"ו ממטבע ו' הם ח' פשו' ממטבע ג' כי ו' כפל ג' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה בין שניהם כ"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|
וידוע כי ערך ג' אל ד' ג' רביעיות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
על כן יהיה חילוף י"ו פשו' ממטבע ד' הם י"ב פשו' ממטבע ג' והנו דינר אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וג' דינ' ממטבע האחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| וככה תוכל להשיב חלוף מה שלקח לאיזה מטבע שתרצה ואין צורך להאריך | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Another example, which is difficult because it has difficult ratios: | דמיון אחר קשה כי אין לו ערכים כי אם בקושי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The moneychanger has three [kinds of] coins. One zahuv is worth five dinar of the first [kind of coins]; or seven of the second [kind]; or nine of the third [kind]. [A man] brings one zahuv and wants to take from all [kinds of coins] for one zahuv equally.
|
וזהו מחליף יש לו ג' מטבעים האחד ה' דינ' בזהוב והשני ז' והשלישי ט' והביא זהוב ורצה לקחת בשוה מספר שוה מכלם בזהוב אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עשה כמשפט וכפול ה' בז' והעולה ל"ה וכפול זה על ט' יהיו שט"ו והוא המורה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וחמישיתו ס"ג ושביעיתו מ"ה ותשיעיתו ל"ה והנה כשנחבר אלה שלשתם יהיו קמ"ג והוא הדינ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נחלק המורה על זה המספר יהיו ב' דינ' וישארו כ"ט חלקים מן קמ"ג וככה יקח מכל מטבע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולבחון זה קשה הוא כי אם על הדרך שאומר לך בעבור החלקים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה נחל לבחון להשיב המספר הנזכר ממנו ממטבע ז' וממטבע ט' אל מטבע ה' וככה נעשה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נשיב הדינ' חלקי' מקמ"ג ונשים עמהן החלקים שהם שט"ו והוא המורה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונבקש להחליף מטבע ז' אל ה' ונכפול שט"ו על ה' ויהיו אלף תקע"ה נחלקם על ז' יהיו רכ"ה חלקים ממטבע ה' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
גם אלף תקע"ה על ט' לדעת כמה יהיו מהמטבע השני והנה קע"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר תחבר אלה ג' מספרים שהם ממטבע אחד שט"ו גם רכ"ה גם קע"ה והנה הכל תשט"ו חלקנום על קמ"ג שהוא הדינ' [51]עלו ה' דינ' שוים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
או אם תרצה חשוב ככה כבר היו ממטבע ה' ב' דינ' וכ"ט חלקים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר החלפנו זה המספר ממטבע ז' יהיו רכ"ה חלקים שהם דינ' אחד ופ"ב חלקים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם ממטבע ט' יהיו קע"ה שהם דינ' אחד ול"ב חלקים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חברנו כל החלקים יהיו קמ"ג שהם דינ' אחד והנה המספר אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועוד נשיב הכל למטבע ז' והנה כבר היו לנו שט"ו חלקים שהוא המורה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונרצה לדעת כמה חלקי' יהיו ממטבע ה' והנה נכפול שט"ו על ז' יעלו אלפים ור"ה נחלק על ה' יעלו תמ"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
גם נחלק עוד זה המספר על ט' יהיה העולה רמ"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נחבר כל אלה החלקים ונחלק המחובר על קמ"ג יהיו ז' דינ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועוד נשיב הכל למטבע ט' והנה יש לו מהמטבע שלו שט"ו חלקים שהוא המורה שהם ב' דינ' גם כ"ט חלקי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונשוב לכפול שט"ו על ט' יהיו אלפים ותתל"ה נחלק זה המספר על ה' יהיו תקס"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
גם נחלק זה על ז' שהוא המטבע האחר יהיו ת"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר נחבר אלה החלקים של ג' מטבעים יהיו אלף רפ"ז וכאשר נחלק זה על קמ"ג יהיו ט' דינ' שלמים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Do the same if there are [4 types] of coins or more. | וככה תעשה אם היו מטבעים או יותר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| explanation - the position of the zero [= representing the unknown] | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Now I want to explain to you where to put the wheel. So we shall give an example: | עתה ארצה לבאר לך באיזה מקום תשים הגלגל והנה נעשה דמיון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Payment Problem | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Question: Reuven hired Shimon to work with him for 17 days and he will pay him 11 pešuṭim, but he worked 9 days.
|
שאלה ראובן שכר שמעון שיעבוד עמו י"ז ימים ויתן לו י"א פשו' והנה עבד ט' ימים[note 87]
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והוא אין ספק כי כערך הימים שעבד אל כל הימים שהיה התנאי שאותו הערך בעצמו יקח מי"א שהם הפשוטים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה נשים הגלגל הראשון והשני י"א והשלישי ט' והרביעי י"ז ככה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם נרצה נשים הגלגל שני ומספר הרביעי ט' שנעשה ככה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כי כאשר שמנו המספר הגדול בראשונה של פשוטים ככה נשים במספר השני המספר הגדול של ימי העבודה שלישי ראשון לשנים האחרונים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונוכל לשום הגלגל שלישי כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונוכל לשום הגלגל רביעי ככה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והכל שוה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Payment Problem - Carrying Wheat | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Question: Reuven hired Shimon to carry for him 13 measures of wheat on his beast a path of 17 miles and his payment will be 19 pešiṭim, but he carried 7 measures for a distance of 11 miles. How much should be his wages?
|
שאלה ראובן שכר שמעון שיוליך לו על בהמתו י"ג מדות חטה מהלך י"ז מילין ויהיה שכרו י"ט פשוטים והוא הוליך שבע מדות מהלך י"א מילין כמה שכרו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ככה תעשה צריך אתה לעשות הערכים פעמים כי אין דרך אחרת להוציאם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וחשוב כי הז' מדות הוליך אותם כל התנאי שהם י"ז והנה תעשה ככה הדמיון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה נכפול הקצוות שהם ז' וי"ט יהיו קל"ג נחלקם על י"ג יעלו י' שלמים ונשארו ג' חלקים מי"ג בפשוט אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ובעבור שלא הוליך אלא ז' מדות רק י"א מילין אנחנו צריכים לעשות ערך אחר ודמיון אחר ונעשה ככה י"א י"ז גלגל י' גם ג' חלקים מי"ג וזה דמיונו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ובעבור שאנו צריכין לכפול הקצוות ולחלק העולה על י"ז ויש לנו ברביעי ג' חלקים מי"ג צריכין אנו לבקש מורה אחד לשניהם וככה נמצאנו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שנכפול י"ג על י"ז יעלו רכ"א והוא המורה והוא אחד שלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה ג' חלקים מי"ג יהיו נ"א מרכ"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונשוב לכפול י"א על י' יהיו ק"י גם נכפול י"א שהם שלמים על נ"א שהם חלקים ויעלו תקס"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Payment Problem - Digging a Hole | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Question: Reuven hired Shimon to dig for him in the ground 10 in length, 10 in width, for which he would pay him 17 pešuṭim, but Shimon dug 5 in length and 5 in width | שאלה ראובן שכר שמעון שיחפור לו בקרקע י' באורך וי' ברוחב ויתן לו י"ז פשו' ושמעון חפר ה' באורך וה' ברוחב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אין ספק כי הרביעית יקח כי כפל חצי על חצי רביעי אחד שלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כי אלו היה כופל חצי האורך על כל הרוחב או חצי הרוחב על כל האורך אז היה לוקח חצי הממון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If he said that he agreed with him that he should dig 7 in length, 6 in width, 5 in depth, and for this he would pay him 11 pešiṭim, but he dug 6 in length, 5 in width, 4 in depth. How much should be his payment?
|
רק אם אמר שהסכים עמו שיחפור ז' באורך וו' ברוחב וה' בעומק ויתן לו י"א פשו' והוא חפר ו' באורך וה' ברוחב וד' בעומק כמה שכרו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה אנחנו צריכים לערכים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונכפול המספר הראשון שהוא ז' על ו' הרי מ"ב כפלה על ה' שהוא העומק ויהיו ר"י | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
גם נכפול המספר השני שהוא ו' על ה' והם ל' גם נכפול זה על ד' שהוא העומק יהיו ק"כ | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועתה נעשה דמיון הערכים כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפלנו האמצעיים הידועים והיו אלף וש"כ חלקנום על ר"י עלו ו' שלמים ונשארו ס' שהם שתי שביעיות פשוט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Pricing Problem - Find the Amount | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Question: a man sells 13 measures of wheat for 23 pešuṭim. How many measures will he sell for 7 [pešuṭim]?
|
שאלה אדם מוכר חטה י"ג מדות בכ"ג פשו' כמה מדות יתן בז' פשו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נעשה דמיון הערך כמשפט הזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה כפול הקצוות שהם נודעים יהיו צ"א נחלקם על כ"ג יהיו ג' מדות וכ"ב חלקים מכ"ג במדה אחת | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Pricing Problem - Find the Price | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| We reverse the question, by that we want to know how much will he get for 7 measures?
|
ועוד נהפוך הענין שנבקש לדעת בכמה יתן ז' מדות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה נעשה הדמיון כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נכפול הקצוות יהיו קס"א נחלקם על י"ג יהיו י"ב פשו' וה' חלקים מי"ג בפשו' אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Motion Problem – Pursuit | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Question: a man sends a messenger to walk 29 miles a day. After 10 days, he sends another messenger to walk after him 37 miles a day. When will he catch up with him?
|
שאלה אדם שלח רץ שילך בכל יום כ"ט מלין ואחר עשרה ימים שלח רץ אחר אחריו שילך בכל יום יום ל"ז מילין מתי ישיגנו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נכפול המילין שהלך בי' ימים יהיו ר"צ נחלקם על היתרון שבין שני המהלכים שהוא ח' והנו ל"ו ימים ורביעית יום | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Give and Take Problem | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Question: a man left his city and arrived to another country. He took an oath that if God will double his money he will donate two pešuṭim each day. After two days he ran out of money. How much did he bring?
|
שאלה אדם יצא מעירו ונכנס במדינה אחרת נדר אם יכפול המקום ממונו יתן בכל יום ב' פשו' לסוף ד' ימים הלך ממונו כמה הביא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
האמת כי היה לו ב' פשוטים פחות שמינית פשוט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Motion Problem – Encounter | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Question: Reuven left his city on the morning of the first day of the month, to meet his brother Shimon in his city. On that same day Shimon left his city, to meet his brother Reuven in his city. The distance between the two cities is 100 miles. Reuven walks 19 miles a day and Shimon walks 17 miles a day. We ask: when will they meet? | שאלה ראובן יצא מעירו ללכת לקראת שמעון אחיו לעירו בקר יום ראשון של ראש החדש ובעצם היום הזה יצא גם שמעון מעירו ללכת לקראת ראובן אחיו לעירו והמרחק בין שני הערים ק' מילין ומהלך ראובן ביום אחד י"ט מילין ומהלך שמעון ביום אחד י"ז מילין נשאל מתי יתחברו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ככה תעשה חבר שני המהלכים והם ל"ו חלק עליו המאה מילין יהיה שני ימים ישארו כ"ח חלקים מל"ו ביום אחד שהם ז' תשיעיות יום | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותוכל להושיבם לשעות היום בדרך הערכין | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וככה תעשה[52] כפלנו ז' על י"ב עלו פ"ד חלקנו על ט' עלו ט' והם שעות נשארו ג' שהם שלישית שעה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועל דרך אחרת ידענו כי ערך י"ב אל ט' כמוהו ושלישיתו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כי תשיעיות היו לנו והנה נקח לז' תשיעיות ז' שלישיות נחלקם על ג' יהיו ב' שעות ושלישית שעה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם בקשנו לדעת כמה מילין הלך ראובן | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כבר ידענו כי בשני ימים הלך ל"ח מילין | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכבר אמרנו כי ז' תשיעיות היום הלך והנה נעשה הערך כך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפלנו ז' על י"ט עלו קל"ג חלקנום על ט' עלו י"ד ושבע תשיעיות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה הכל נ"ב מילין וז' תשיעיות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Payment Problem - three workers, three different daily wages, the same actual payment | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Question: A man hired three brothers – Reuven, Shimon, and Levi – to do his work for 20 days from morning until evening, any one of them in turns so that the work will not cease. If Reuven works all the days he will pay him 5 zehuvim; if Shimon – 4, if Levi – 3. They worked together for the 20 days and there was a a supervisor sitting with them, who wrote down how many hours and parts of hours each of them worked a day. Finally, he paid each of them an equal share. We wish to know: how much is the share of each of them?
|
שאלה אדם שכר ג' אחים ראובן שמעון ולוי שיעשו עבודתו כ' ימים מן הבקר עד הערב מאיזה מהם שתהיה ולא תשבות המלאכה והנה אם עבד ראובן כל הימים יתן לו ה' זהובים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דע כי ראובן ישמש ד' ימים בזהוב אחד ושמעון ה' ימים ולוי ו' ימים וב' שלישיות יום והנה הכל ט"ו שלמים וב' שלישיות יום אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נחלק כ' על זה המספר ועלה אחד שלם ונשארו ד' שלמים ושלישית אחד והנה בעבור השלישית נשיב הכל שלישיות והנה נשיב הכ' יום ס' שלישיות והט"ו וב' שלישיות מ"ז שלישיות והד' ושלישית יום י"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועתה נבקש כמה חיוב כל אחד שיעבוד עד שישלימו הכ' יום | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה נחל מלוי שהוא חייב לשמש בזהוב שלקח ו' ימים וב' שלישיות יום ונעשה מאלה שלישיות ויהיו כ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונבקש לדעת כמה יש לו לעבוד בעבור י"ג פשו' שלקח ונעשה הערך כך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפלנו י"ג על כ' והיו ר"ס חלקנום על מ"ז עלו ה' ונשארו כ"ה חלקים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונבקש לדעת כמה עבד שמעון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה חייב לעבוד בעבור הזהוב ה' ימים שהם ט"ו שלישיות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונבקש לדעת כמה יעבוד בעבור י"ג פשו' שלקח והנה נעשה הערך כך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נכפול ט"ו על י"ג יעלו קצ"ה נחלקם על מ"ז עלו ד' ונשארו ז' חלקים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונבקש לדעת כמה עבד ראובן | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה עבד בשביל הזהוב ד' ימים שהם י"ב שלישיות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונעשה בעבור הי"ג פשוטים שלקח הערך כך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נכפול י"ג על י"ב יעלו קנ"ו נחלקם על מ"ז עלו ג' ונשארו ט"ו חלקים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר תחבר אלה החלקים יתחבר מהם שעה אחת בלי תוספת ומגרעת | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר תחבר זאת השעה אל השעות הנזכרות יהיו י"ב שעות שהוא יום אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר תחבר היום לימים הנזכרים יהיו עשרים יום בלי תוספת ומגרעת | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Boiling Problem | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Question: A man had 10 measures of must and he wants to cook them so that only one-third remains. He starts to cook [them] until eight measures are left of them. Then, two measures overflow. Now he wants to cook [the remaining 6 measures] until it is reduced [as he planned] at first.
|
שאלה אדם היו לו י' מדות מתירוש ורצה לבשלם עד שלא ישאר מהם כי אם השלישית והנה החל לבשל עד שנשארו מהם ח' מדות ונשפך ב' מדות והנה ירצה לבשלם עד שיהיו כמשפט הראשון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה יש לך ג' מספרים ידועים הא' כמה שלישית י' ידוע כי הוא ג' ושליש וידוע כי שמנה יהיו המדות שיתבשלו וידוע כי ששה נשארו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה תעשה הדמיון ככה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה נכפול ו' על ג' ושליש יהיו כ' נחלקם על ח' יהיו שנים וחצי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Another example: We have 9 measures of must, and he wants them to be cooked until the third part of it remains. It was now cooked until 6 measures were left. Then 4 measures were overflow, and 2 measures remain. | דמיון אחר היו לנו ט' מדות תירוש וירצה שיתבשלו עד שישאר השליש והוא נתבשל עד שנשארו ו' מדות ונשפכו ד' וב' נשארו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וככה הערך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפול ב' על ג' עלו ו' יהיה אחד והנה המשפט להיות המבושל מדה אחת | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| How Much Problem - Money | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Question: an amount of money, we sum its fifth, its seventh, and its ninth and they are 10, how much is the money?
|
שאלה ממון חברנו חמישיתו ושביעיתו ותשיעיתו והיו עשרה כמה הממון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נבקש המורה והוא שט"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והחלקים הנזכרים הם קמ"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כאשר תחלק שט"ו על ה' יעלו ס"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכשתחלק על ז' יעלו מ"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועל ט' יעלו ל"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חברם יחד יעלו קמ"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונעשה הערך כך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נכפול שט"ו על י' יעלו ג' אלפים וק"נ נחלקם על קמ"ג עלו כ"ב שלמים וד' חלקים מקמ"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| First from Last Problem - Money | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| We do the opposite: An amount of money - we have subtracted from it its fifth, its seventh and its ninth and 10 remain.
|
נעשה להפך ממון חסרנו ממנו חמישיתו שביעיתו ותשיעיתו ונשארו י' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נחסר קמ"ג שהם השברים מן שט"ו שהוא המורה ישארו קע"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונעשה הערך כך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפלנו י' על שט"ו עלו ג' אלפים וק"נ חלקנום על קע"ב עלו י"ח שלמים ונ"ד חלקים מקע"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
לקחנו חמישית ושביעית ותשיעית זה המספר ישארו לנו י' שלמים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועל זה הדרך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Find a Quantity Problem - Whole from Parts - Tree | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Question: A tree, a third of it is in the water, a quarter of it is [ingrained] in the soil, and 10 cubits of it are up above the water, how much is the length of the whole tree? |
שאלת האילן ששלישיתו במים ורביעיתו בעפר ולמעלה מן המים י' אמות כמה גבהות כל האילן | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
Common Denominator: We seek for a number that has a third and a quarter; it is 12. |
נבקש מנין שיש לו שלישית ורביעית והוא י"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ושלישיתו ורביעיתו מחוברים ז' נחסרם מי"ב ישארו ה' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נעשה הערך כך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה כפלנו הקצוות עלו ק"כ חלקנום על ה' עלו כ"ד וזהו גבהות כל האילן | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כי שלישיתו שמנה ורביעיתו ששה והמחוברים י"ד נחסרם מכ"ד ישארו י' שלמים לא פחות ולא יותר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Another example: A tree, a seventh of it is in the water, a ninth of it is [ingrained] in the soil, and 8 [cubits] of it are up above the water.
|
דמיון אחר אילן שביעיתו במים ותשיעיתו בעפר ולמעלה מן המים ח' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה המורה ס"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נחסר ממנו י"ו שהוא השביעית והתשיעית נשארו מ"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונעשה הערך כך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה כפלנו הקצוות והיו תק"ד חלקנום על מ"ז עלו י' שלמים גם ל"ד חלקים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ושביעית זה המספר אחד שלם וכ"ה חלקים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותשיעיתו אחד שלם וט' חלקים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חברנו החלקים הנזכרים והם ל"ד והשלמים ב' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חסרנום מהמספר הנזכר נשארו ח' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Divide a Quantity Problem - Proportional Division – Inheritance | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Question: Jacob died. His son Reuven issued a deed with two credible witnesses, according to which his father Jacob has given him alone all the property he had and instructed so in case of death. His son Shimon issued a deed as well, according to which his father instructed that half of his property should be granted to him. Levi also issued a deed, according to which his father instructed that one-third of his property should be given to him. Yehudah too issued a deed, according to which one-quarter of his property should be granted to him. All of them wrote this in Jerusalem in the same day, the same time, the same hour | שאלה יעקב מת והוציא ראובן בנו שטר בשני עדים כשרים שנתן לו לבדו יעקב אביו כל הממון שהיה לו וצוה כן מחמת מיתה ביום מותו גם הוציא שמעון בנו שטר שאביו צוה מחמת מיתה שינתן לו חצי ממונו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Three methods to divide the property between the four sons each according to his relative portion: | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה חכמי ישראל מחלקים אותו על דרך בקשת כל אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וחכמי הגוים על דרך ערך הממון שלכל אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וחכמי החשבון יחשבו כי הממון היה אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר תחבר אליו חציו ושלישיתו ורביעיתו יהיה הכל שנים וחצי ששית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה נשים האחד שלם ששים שיש לו כל החלקים הנזכרים והנה יהיה בין הכל קכ"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
או נשים האחד שלם י"ב והשברים הנזכרים י"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ושוה יצא המספר באחרונה איזה מהם שתקח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה נבקש כמה יקח ראובן כפי ערך ממונו ונעשה הערך ככה על דרך ששים כי הוא מבקש כל הממון ונאמר כי הממון עשרה דינרים שהם ק"כ פשוטים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה תורת ערך הממון שיקח ראובן | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפלנו הקצוות ועלו ז' אלפים ור' חלקנום על קכ"ה עלו נ"ז פשו' וע"ה חלקים וזה חלק ראובן | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וזו צורת ערך שמעון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נכפול הקצוות ונחלק כמשפט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וזו צורת חלק לוי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נכפול ונחלק כמשפט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וזו צורת חלק יהודה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Another method in a shorter way [according to the Gentile sages]: | ענין אחר בדרך קצרה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
יקח שמעון חצי חלק ראובן | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
גם יקח לוי שליש חלק ראובן | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
גם יקח יהודה רביעית חלק ראובן | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר תחבר כל אלה החלקים והשלמים יהיו ק"כ פשו' שהם י' דינ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| According to the procedure of the sages of Israel: | ועל דרך חכמי ישראל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
יאמרו הג' אחים הגדולים ליהודה אחיהם אין אתה מערער רק על ל' פשוטים וערעור כל אחד ממנו שוה בהם קח ז' וחצי שהוא הרביעית ולך מעמנו וכמו כן יקח כל אחד מהג' אחים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועוד יאמר ראובן ללוי אין אתה מערער רק על מ' פשו' וכבר לקחת חלקך מהל' שארבעתנו ערערנו עליהם קח אתה שלישית י' שהוא רביעית מ' ולך מעמנו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
גם יאמר ראובן לשמעון אין אתה מערער אלא על חצי הממון שהוא ס' והחצי האחר הוא כלו שלי וכבר לקחת חלקך מהמ' והנה נשאר ביני ובינך הערעור בעשרים קח חציים ולך מעלי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וחלק ראובן שמונים גם חמש ששיות פשו' אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר תחבר אלו החלקים יהיו עשרה דינ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Rule of Four in Astronomical Tables |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Now, I shall explain the method of the astrologers in the tables of the determination of the planets. | ועתה אפרש דרך חכמי המזלות בספרי הלוחות בתקון המשרתים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| When determining the moon and also when determining 5 planets, there is a row that is called the row of ratio and this ratio is to 60. | כי בתקון הלבנה גם בתקון ה' המשרתים טור יקרא טור הערך וזה הערך הוא אל ס' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| For, if 60 is written in the row of the ratio, | כי אם היה כתוב בטור הערך ס' יהיה בטור אחריו שיקרא הטור החמשי או הכתוב בטור השביעי מה שיהיה כתוב באחד מהם ראשונים לבדם או ראשונים ומעלות הכל יקח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| So it is when determining the position of the moon. | וככה בתקון מקום הלבנה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If it is less than 60, one should take the ratio from the parts that are written in the fifth or seventh row. | ואם היתה פחותה מס' יקח כפי הערך מהחלקים הכתובים בטור החמישי או השביעי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון יש חלקים יתרים על המעלות מ' ובטור הערך ט"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואתה צריך לכפול ט"ו על מ' והעולה תחלקנו על ס' והוא המבוקש ועשה דמיון הערך כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפול ט"ו על מ' עלו ו' מאות חלקם על ס' יעלו י' חלקים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ויותר קרוב מזה שתבקש מה ערך מ' אל ס' והם ב' שלישיות גם זה הערך קח מט"ו והנו י' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
או בקש מה ערך ט"ו אל ס' והנו רביעית ס' קח רביעית מ' והוא י' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון אחר המספר האחד ל' והשני מ"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה ערך מ"ה אל ס' ג' רביעיות נקח שלש רביעיות ל' והם כ"ב וחצי שהם ל' שניים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
או נקח הערך מן הל' שהוא חצי ס' ג"כ נקח חצי מ"ה והנו כ"ב וחצי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון לב' מספרי' שהאחד יש לו ערך והשני אין לו ערך כמו המספר האחד כ' והשני ל"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה ערך כ' אל ס' שלישית והנה נקח שלישית ל"ג והם י"א ראשונים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וככה יבא בדרך הכפל כאשר תכפול כ' על ל"ג ותחלק העולה על ס' והעולה בחלוק יהיה י"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ובעבור כי ל"ג קרוב מחצי ס' נקח גם הערך ממנו והנו י' ראשונים שהוא חצי כ' ובעבור שיש לנו תוספת ג' על החצי נכפול ג' על כ' יהיו ס' ואין ספק כי הם שניים כי כפל ראשונים על ראשונים יעלו שניים כי אחד על אחד שנים והם ס' שניים והם חלק ראשון נחברם על י' והוא י"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון אחר המספר האחד כ' והשני כ"ח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה ערך כ' אל ס' שלישית ושלישית כ"ח ט' ראשונים וב' שניים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועוד נחשוב כי כ"ח חצי ס' כי הוא קרוב ממנו והנה חצי כ' י' ובעבור כי ב' יחסרו מהחצי נכפול ב' על כ' יהיו מ' שניים נחסרם מהראשון אחד יהיו הנשארים ט' ראשונים וכ' שניים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון לחסר הערך שחשבונו האחד י"ד והשני כ"ט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה נקח הערך מכ"ט ונחשוב כי הוא חצי ס' נקח חצי י"ד והם ז' ראשונים ובעבור כי אחד יחסר מהחצי נכפלנו על י"ד יהיו י"ד שניים נחסרם מחלק ראשון ישארו ו' ראשונים ומ"ו שניים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון אחר המספר האחד לחסר והשני להוסיף והנה נשים האחד י"ח והשני מ"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה נקח הערך ממ"ב ונחשוב כי הוא שתי שלישיות נקח שתי שלישיות י"ח והנה י"ב ובעבור שיש לנו תוספת ב' נכפלם על י"ח יהיו ל"ו שניים וככה יהיה העולה בדרך הכפל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וגם נקח הערך מי"ח ונחשוב כי הוא שלישית והנה שלישית מ"ב י"ד ובעבור שהוספנו ב' נכפלם על מ"ב והיו פ"ד שניים שהם חלק ראשון וכ"ד שניים נחסרם מי"ד ישאר כמספר הראשון שהוא י"ב גם ל"ו שניים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונוכל לקחת עוד הערך בתוספת משניהם שנחשוב י"ח שהוא רביעית ס' והנה נקח רביעית מ"ב יהיו י' ראשונים ול' שניים ובעבור שיש לנו תוספת ג' נכפול מ"ב על ג' יהיו קכ"ו שניים שהם ב' ראשונים וו' שניים נוסיף זה על המספר הנזכר יהיו י"ב ראשונים ל"ו שניים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואילו היינו חושבים כי מ"ב הם ג' רביעיות היה הדבר יוצא נכון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונוכל לעשות הערך מי"ד שנחשוב כי הוא רביעית ס' נקח רביעית כ"ט והם ז' ורביעית שהם שניים ובעבור כי החסרון מרביעית הוא חלק ראשון נכפול כ"ט על כ"ט יהיו כ"ט שניים נחסרנו מן המספר הנזכר ישארו ו' ראשונים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| I shall tell you the rule: if the parts in the row of the ratio do not share a ratio [to 60], proceed in the way of the multiplication, as I have mentioned for the fraction. | וכלל אומר לך כי אם לא היה ערך לחלקים הנמצאים בטור הערך שוב לעשות על דרך הכפל כאשר הזכרתי בשברים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Always see: if there are any fractions added to the degrees of the determined center that are less than 30, leave them out and take the minutes that are written in the row of the ratio corresponding to the preceding degrees. | ולעולם ראה אם היו חלקים נוספים על מעלות המוצק המתוקן והם פחותים מל' הניחם וקח הראשונים הכתובים בטור הערך כנגד המעלות שעברו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If the fractions that are with the determined center are more than 30, add one degree to the preceding degrees and take the fractions you find corresponding to it in the row of the ratio, whether it is after the whole degree or higher. | ואם החלקים שהם עם המוצק המתוקן יותר מל' הוסף מעלה אחת על המעלות שעברו וקח מה שתמצא כנגדה בחלקי טור הערך בין שתהיה אחר המעלה השלמה או למעלה ממנה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If you find the determined quotient between 4 constellations to 8 constellations and you find that there is a difference between the fractions of the row of the ratio corresponding to the preceding degree and the fractions of the row of the ratio corresponding to the added degree - the difference between them will always be only one minute at most - proceed in the way of the multiplication, that you give the fractions added to the preceding degree the seconds they deserve. | ואם מצאת המנה המתוקנת שהיא בין ד' מזלות עד ח' מזלות ומצאת כי יש הפרש בין חלקי טור הערך שהם כנגד המעלה שעברה ובין חלקי טור הערך שכנגד המעלה הנוספת ולא יהיה לעולם ביניהם רק חלק ראשון עשה כדרך הכפל שתתן לחלקים הנוספים על המעלה שעברה מה שראוי מהשניים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| You need this method when determining the position of Mars or Mercury, however you do not need it for the determination of the position of Saturn and Jupiter, because in the fifth row and the seventh row there are no degrees, only minutes. | וזה המעשה יש לך צורך בתקון מאדים או כוכב חמה רק בתקון שבתי וצדק אין לך צורך כי אם בטור החמישי גם בטור הז' אין מעלות כי אם ראשונים לבדם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Chapter Seven - Extraction of Roots |
השער הז' בהוצאת השרשים | ||||||||
| All the numbers are according to three ways: | כל המספרים הם על שלשה דרכים | ||||||||
|
האחד שרשים והשני מרובעים והשלישי לא שרשים ולא מרובעים | ||||||||
|
והנה המרובע הוא המחובר מכפל שורש על עצמו | ||||||||
|
כמו ט' כי הוא מרובע ארכו כרחבו ושרשו ג' | ||||||||
| There are numbers that have no true [rational] root at all, and they are the majority. | ויש חשבון שאין לו שורש אמת כלל והוא הרוב | ||||||||
|
כמו ב' במעלה הראשונה גם ג' וה' וו' וז' וח' | ||||||||
| The square of an integer is always greater than the root. | ולעולם מרובע השלמים גדול מהשורש | ||||||||
| Vice versa for the squares of fractions. | והפך הדבר במרובעי' הנשברי' | ||||||||
| This is because when multiplying a fraction by a fraction, the product is less than the multiplied fraction. | והיה כן בעבור כי כפל שבר על שבר יהיה המחובר פחות מהשבר הנכפל | ||||||||
| The one alone is both root and square; because it is between the fractions and the integers. | והיה האחד לבדו שורש ומרובע כי הוא בין השברי' והשלמי' | ||||||||
Integers |
|||||||||
| First, we shall give scales [= test methods]: | והנה נתן בתחלה מאזנים | ||||||||
Observe:
|
הסתכל אם לא היו מאזני המרובע ככפל מאזני השרש על עצמו אין המספר מרובע | ||||||||
|
ואם היה כמוהו יתכן להיות מרובע | ||||||||
|
דמיון המרובע קמ"ד | ||||||||
|
והמאזנים ט' והשרש י"ב ומאזניו ג' וכפל על עצמו הוא ט' | ||||||||
| והוא מאזני השרש ת' | |||||||||
| Other scales: | מאזנים אחרים | ||||||||
|
אם מאזני המספר ב' או ג' או ה' או ו' או ח' איננו מרובע | ||||||||
|
ואם היו המאזנים אחד מהמרובעים שהם במעלה הראשונה שהם א' או ד' או ט' גם ז' עמהם יתכן להיות מרובע | ||||||||
| Other scales: | מאזנים אחרים | ||||||||
|
אם היה במספר המבוקש ממספרי המעלה הראשונה ב' או ג' או ז' או ח' אין המספר מרובע | ||||||||
|
ואם היה אחד מן המרובעים א' או ד' או ט' או מן המתגלגלים שהם ה' ו' יתכן שיהיה המספר מרובע | ||||||||
| Other scales that are reliable: | מאזנים אחרים שהם מאזני צדק | ||||||||
|
אם מצאת במספר המבוקש מן המעלה הראשונה אחד דע שיש בשורש אחד או ט' | ||||||||
|
ואם היה במספר ד' דע שיש בשורש ב' או ח' | ||||||||
|
ואם היה במספר ו' דע שיש בשרש ד' או ו' | ||||||||
|
ואם במספר ט' דע כי יש בשרש ג' או ז' | ||||||||
|
ואם במרובע ה' דע שיש בשרש ה' | ||||||||
| Now I shall give you a way by which you can know which of the two mentioned [digits] is in the root. | ועתה אתן לך דרך שתוכל לדעת איזה מן השנים הנזכרים יהיה בשרש | ||||||||
| Now, note that the square numbers that are in the first rank are 3, which are 1, 4 9, | ועתה שים לבך כי המרובעים במעלה הראשונה הם ג' והם א' ד' ט' | ||||||||
| In the second rank they are 6, which are 16, 25, 36, 49, 64, 81. | ובמעלה השנית ו' והם י"ו כ"ה ל"ו מ"ט ס"ד פ"א | ||||||||
| All the ranks that follow these two have the same method: | וכל המעלות שהם אחר אלה השתים דרך אחת להן | ||||||||
|
כי כל מעלה שאיננה זוג הולכת על דרך המעלה הראשונה | ||||||||
|
וכל מעלה שהיא זוג הולכת על דרך המעלה השנית | ||||||||
| The square numbers that are analogous to the square numbers, which are in the first rank, are always one digit, and those that are in the second rank as well as every even [rank] are two digits and so are all the analogous. | ולעולם יהיו המרובעי' הנמשלים למרובעים שהם במעלה הראשונה מספר אחד ואשר הם במעלה השנית וכל זוג הם שני מספרים גם כל הנמשלים | ||||||||
| From the analogous squares you can know all the square numbers that precede them or succeed them. | ומהנמשלים תוכל לדעת כל המרובעים שהם לפניהם או לאחריהם | ||||||||
| When you know the root of the first rank or the second and you wish to find the root of the analogous number in whichever rank, proceed as follows: | וכאשר תדע שרש המעלה הראשונה או השנית ותרצה לדעת שרש הנמשל באיזו מעלה שיהיה ככה תעשה | ||||||||
| Know that the units that are in the first rank, are tens in the third rank, hundreds in the fifth, thousands in the seventh, tens of thousands in the ninth, hundreds of thousands in the eleventh, and so on by skipping endlessly. | דע כי ההוה במעלה הראשונה מהאחדים הם במעלה השלישית עשרות ובחמישית מאות ובשביעית אלפים ובתשיעית עשרת אלפים ובאחד עשר מאת אלף וככה עד אין קץ בדלוג | ||||||||
| Because, the skipping is from an odd number to an odd number. | כי ידלג ממספר שאיננו זוג אל מספר שאיננו זוג | ||||||||
| The units that are in the second rank of the roots are tens for the fourth rank, hundreds for the sixth, thousand for the eighth, tens of thousands for the tenth and hundreds of thousands for the twelfth. | והאחדים שהם במעלה השנית בשרשים הם מעלה הרביעית עשרות ובששית מאות ובשמינית אלפים ובעשירית עשרת אלפים ובשנים עשר מאה אלף | ||||||||
| Because, the skipping is always from an even number to an even number. | כי לעולם ידלג מזוג אל זוג | ||||||||
| Now I will tell you how to proceed if you know the analogous square and you know its root: | ועתה אומר לך איך תעשה כאשר תדע המרובע הנמשל ותדע שרשו | ||||||||
Subtract the square from the sought number, after making sure that you take only the preceding square, which is the closest to the number, see the difference between the square [and the sought number] and divide it by double the root of the preceding square. Make it so that you do not give everything you can for it, but leave a little bit of it so that you can still take the square of the quotient of it. When you see that the difference between the preceding square [and the sought number] is as much as the quotient, then you know that the square number is true.
|
חסר המרובע מהמספר המבוקש אחר שתשמור שלא תקח לעולם כי אם המרבע שעבר שהוא קרוב אל מספר וראה המרחק שבין המרבע וחלקהו על כפל שרש המרבע שעבר וככה עשה שלא תתן לו כל מה שתוכל רק הנח ממנו שתוכל לקחת מרבע מה שעלה בחלוק וכאשר תראה שיהיה המרחק בין המרובע שעבר כמספר מה שעלה בחלוק אז תדע שהמרבע אמת | ||||||||
If the sought number is less than the analogous square number, see how much the difference between your number and the succeeding square number is. Since you have to give it a little bit more than you can because your number precedes the analogous squares, as I will explain later, do not add the square of the quotient to your number. See if the number is as much as double the root multiplied, then you know that your number is true.
|
ואם היה המספר המבוקש פחות מהמרבע הנמשל ראה כמה מרחק בין מספרך ובין המרבע הבא לפי שאתה צריך לתן לו מעט יותר ממה שתוכל בעבור היות מספרך לפני המרבע הנמשל כאשר אפרש ואל תכניס במספרך מרובע החלוק | ||||||||
| If it is 1, subtract one from tens, then 9 remain. | כי אם היה אחד חסר מן העשרות אחד וישארו ט' | ||||||||
| In this way you can know: if you find 1 or 9 in the square, then it must be in the root, as you will see from the examples. | ובדרך הזה תוכל לדעת כשתמצא א' במרבע או ט' ראוי להיות בשרש כאשר תראה בדמיונות | ||||||||
|
דמיון בקשנו לדעת מרובע שעבר קרוב אל מאתים | ||||||||
|
והנה זה מהמעלה השלישית שאיננה זוג ונבקש זה מהמעלה הראשונה וכבר אמרנו כי המרבעי' שיש בה א' ד' ט' והנמשלי' אליהם מאה וד' מאות וט' מאות | ||||||||
|
והנה נתן לו ד' כפולים על כ' הם פ' נשארו כ' נחסר ממנו י"ו שהוא מרובע מה שעלה בחלוק ישאר ד' נחסרנו מהמאתים ישארו קצ"ו וזהו המרבע הקרוב אל מאתים | ||||||||
|
| |||||||||
|
ונוסיף ד' שעלה בחלוק על השרש הראשון שהיה י' יהיו י"ד וזהו שרש המרבע באמת | ||||||||
|
והנה נבחן אותו במאזנים כמשפט ידוע כי מאזני י"ד ה' וכפלו על עצמו כ"ה והנה המאזני' ז' וככה בדרך קצ"ו | ||||||||
|
ובמאזני' אחרי' בעבור שיש שם חשבון מתגלגל נכון להיותו מרבע | ||||||||
|
ובמאזני' אחרי' בעבור שיש במרובעו ו' ראוי להיות בשרש ד' או ו' | ||||||||
|
והנה המרחק מהמרבע הנמשל ק' וכפל [שורש] מרובע הנמשל כ' כפלנוהו על ד' עלו פ' | ||||||||
|
דמיון אחר במעלה הזאת בקשנו לדעת מרבע הקרוב אל ג' מאות | ||||||||
|
וג' מאות יותר קרובי' אל ד' מאות שהוא מרבע הנמשל במעלה הזאת ממרובע הראשון שהוא ק' | ||||||||
|
והנה נסתכל המרחק מהמרבע הבא והוא ק' וידענו כי שרש ד' מאות הוא כ' וכפלו מ' נחלוק ק' עליו ונתן לו מעט יותר ממה שנוכל בעבור היות המספר לפני המרובע | ||||||||
|
וכאשר נכפול ג' על מ' שהוא כפל השרש יהיו ק"כ נחסר זה המספר מת' יהיו ר"פ | ||||||||
|
| |||||||||
|
והנה נחסר הג' מכ' שהוא שרש המרובע הנמשל וישארו י"ז והוא שרש זה המרובע | ||||||||
|
ובעבור כי יש במרובעו ט' הנה התבאר שראוי שיהיה בשרש ז' ולא ג' כי לא יתכן להיות ג' במרובע רק אם היה קרוב אל המרובע הנמשל שעבר | ||||||||
| In this way you can know if there is 1 or 9 in the square according to the distance from the preceding and succeeding analogous squares. | ועל זה הדרך תוכל לדעת במרובע ששם א' או ט' כפי המרחק מהמרובע שעבר ומהמרובע הנמשל העתיד | ||||||||
| Similarly, you can deduce if you find 4 in the square, whether there is 2 or 8 in the square root. | ובזה תוכל להפריש אם מצאת במרובע ד' אם יש בשרש ב' או ח' | ||||||||
| likewise, if there is 6 in the square, you can know whether there must be 4 or 6 in the root. | וככה אם יש במרובע ו' תדע אם ראוי להיות בשרש ד' או ו' | ||||||||
| Now I shall reveal to you the secret why this is so. | והנה אגלה לך קצת זה הסוד למה היה כך | ||||||||
| Know that the two major orbits, one goes east and the other goes west, and that the upper force is one in both. | דע כי השתים המסיבות הגדולות אחת הולכת למזרח ואחת הולכת למערב וכח עליון הוא אחד בשתיהם | ||||||||
| So, one is the square of one, and there is one in the square of 9. | והנה אחד מרובע אחד יש במרובע ט' אחד | ||||||||
| As the number 2 is second to the one, so the number 8 is second to the 9 backwards, since the course of this is opposite to the course of the other. | וכאשר הוא חשבון ב' שני לאחד ככה חשבון ח' שני לט' אחרונית כי מהלך זה הפך מהלך זה | ||||||||
| The square of 2 is 4 and there is 4 in the square of 8. | ומרובע ב' ד' וככה יש במרובע ח' ד' | ||||||||
| The same applies to 3 and 7; 4 and 6. | וכן דרך ג' אל ז' וד' עם ו' | ||||||||
| Thus, the number 5 is left as a mean, therefore it is recurring around itself. | והנה נשאר חשבון ה' אמצעי וע"כ הוא מתגלגל על עצמו | ||||||||
|
ותוכל להוציא המרובע הנז' על הדרך הראשונה שהזכרתי במרובע קצ"ו שתחסר המרובע שהוא ק' מהמספר הנתון שהוא ש' יהיה המספר ר' וידוע כי שרש ק' י' וכפלו כ' והנה לא נוכל לתת לו י' כי לא ישאר מספר שנוכל לחסר ממנו מרובע מה שיעלה בחלוק | ||||||||
|
ונכפול ז' על כ' שהוא כפל השרש יהיו ק"מ נוסיף עליהם הק' שהוא המרובע יהיו ר"מ | ||||||||
|
| |||||||||
|
גם נוסיף שעלה בחלוק על י' שהיה השרש והנה יהיה שרש זה המרובע י"ז | ||||||||
|
דמיון במעלה הרביעית בקשנו לדעת המרובע הקרוב אל אלף ור' | ||||||||
|
והנה בעבור שהוא מספר זוג נבקש במעלה השנית מספר דומה לזה והוא י"ב ומרובע שעבר הוא ט' וככה ט' מאות וכאשר שרש ט' ג' ככה שרש ט' מאות ל' | ||||||||
|
נכפול ס' על ד' יהיו ר"מ נחברם אל המרובע הנמשל שעבר יהיה אלף וק"מ | ||||||||
|
| |||||||||
|
ובעבור שעלה בחלוק ד' נוסיפנו על שרש המרובע הנמשל שעבר שהיה ל' יהיה שרש זה המרובע ל"ד | ||||||||
|
והוא אמת בכל המאזנים | ||||||||
|
דמיון אחר במעלה הזאת נבקש המרבע הקרוב לז' אלפים ת"ק | ||||||||
|
והנה זה המספר דומה לע"ה כי הוא מן הזוגות ובעבור שהוא קרוב אל פ"א יותר מס"ד וידענו כי שרש פ"א ט' וככה יהיה שרש ח' אלפי' וק' צ' וכפלו ק"פ והנה המרחק ו' מאות נתן לו ד' אע"פ שאינו שלם בעבור שהחשבון הנתון הוא לפני המרובע הנמשל | ||||||||
|
וכאשר נכפול כפל השרש על ד' יעלו תש"כ נחסר זה המספר מהמרובע הנמשל יהיה הנשאר ז' אלפים ש"פ | ||||||||
|
| |||||||||
|
ושרשו פ"ו כי נחסר ד' וזהו המרובע באמת | ||||||||
|
וגם אם עשינו בדרך האחרת שהזכרתי בדמיונים שעברו יהיה הדבר שוה | ||||||||
|
דמיון במעלה החמישית נבקש המרובע הקרוב אל כ"ג אלף | ||||||||
|
והנה זה דומה למעלה הראשונה כי איננו זוג והנה י' אלפים כמו אחד וכבר אמרנו מה שהוא במעלה הראשונה אחד יהיה במעלה החמישית מאה והנה המרובע [53]שעבר י' אלפים | ||||||||
|
נוסיפנו על השרש שעבר יהיה ק"נ ונשאר לנו עוד ג' אלפים נחסר ממנו אלפים ת"ק שהוא מרובע מה שעלה בחלוק ישארו ת"ק | ||||||||
|
| |||||||||
|
ויהיה המרובע כ"ב אלף ותת"א והשרש קנ"א | ||||||||
|
כאשר יכפול המספר אם יעלה לעשרות חבר עשרות עם עשרות לפי המעלות ואם יש שם אחדים שים במקום האחדים אשר כפלת | ||||||||
|
דמיון אחר במעלה הזאת נבקש המרובע הקרוב אל פ"ה אלפים | ||||||||
|
והנה הוא קרוב אל המרובע הנמשל שהוא צ' אלף ושרשו ש' והנה המרחק ה' אלפים נחלקנו על כפל השרש שהוא ת"ר ונתן לו ט' הקרוב יותר ממה שנוכל | ||||||||
|
והנה יש לו תוספת ת' נחסרם מהמספר הנתון ישארו פ"ד אלפים ות"ר ועם תוספת פ"א שהוא מרובע מה שעלה בחלוק יהיה פ"ד אלף תרפ"א וזה הוא המרובע באמת | ||||||||
|
| |||||||||
|
ושרשו רצ"א | ||||||||
|
דמיון במעלה הששית בקשנו לדעת המרובע הקרוב אל ר' אלף | ||||||||
|
ובעבור שזאת המעלה מן הזוגות היא דומה לעשרים והמרובע שעבר הוא י"ו וכבר אמרנו כי מה שהוא במעלה השנית אחדים יהיו במעלה הששית מאות והנה השרש ת' והמרובע שעבר ק"ס אלפים והנה המרחק מ' אלף נחלקנו על כפל השרש שהוא ת"ת והנה לא נוכל לתת לו ה' בעבור מרובע החלוק נתן לו ד' שהם מ' | ||||||||
|
ונכפול זה המספר על ת"ת יעלו ל"ב אלף ונשארו ח' אלף נסיר מהם אלף וו' מאות שהוא מרובע מ' ישארו ו' אלף וד' מאות | ||||||||
|
| |||||||||
|
נחסר עוד מרובע ז' נשאר קצ"א נחסר זה המספר מהמספר הנתון ישארו קצ"ט אלף ותת"ט וזהו המרובע | ||||||||
|
| |||||||||
|
והשרש תמ"ז | ||||||||
|
דמיון אחר במעלה הזאת לדעת מרובע הקרוב אל ו' מאות אלף | ||||||||
|
בעבור כי המרובע הנמשל הוא אחרי החשבון נקח המרחק שהוא מ' כי ו' מאות אלף הם כמו ס' וכאשר חלקנום על אלף וו' מאות נתננו לו כל מה שיכולנו ואם יחסר מעט מהאמת | ||||||||
|
| |||||||||
|
חסרנו זה משרש המרובע הנמשל שהוא ח' מאות ושהם דומים לח' והנה השרש תשע"ד וכבר היה לנו מ"א אלף ות"ר והיה לנו מ' אלף שהוא המרחק והנה אין לנו לחסר כי אם אלף וו' מאות מהמספר הנתון ויש לנו להוסיף עליו מרובע כ"ו שעלה בחלוק שהוא תרע"ו | ||||||||
|
| |||||||||
|
דמיון במעלה השביעית בקשנו לדעת המרובע הקרוב אל ה' אלפי אלפים | ||||||||
|
ונאריך לבאר זה הדמיון כהוגן עד שיהיה עקר להוציא המרובעים שהם במעלות אחרות עד אין קץ אע"פ שאין צורך להם בדברי החכמות | ||||||||
|
והנה המעלה הזאת דומה לראשונה והחשבון המבוקש כמו ה' והנה נסיר המרובע שהוא ד' ישאר לנו אלף אלפים והשרש שלנו שעבר אלפים וכפלו ד' אלפים נחלק עליו המספר הנשאר | ||||||||
|
| |||||||||
|
יעלו ת"ת אלף ונשאר לנו ר' אלף נחסר ממנו מרובע מה שעלה בחלוק שהוא מ' אלף נשאר לנו ק"ס אלף | ||||||||
|
| |||||||||
|
ישאר לנו כ"ח אלף נסיר ממנו מרובע מה שעלה בחלוק זה שהוא ל' והם תת"ק נשאר כ"ז אלף וק' | ||||||||
|
| |||||||||
|
נשאר לנו ש"מ נחסר ממנו ל"ו שהוא מרובע ו' ישאר ש"ד | ||||||||
|
| |||||||||
| Test this with all the testing methods and you will find it correct. | ותבחן זה בכל המאזנים ותמצאנו נכון | ||||||||
|
וככה בדרך כפל השרש על עצמו | ||||||||
|
כי כפל אלפים על אלפים יהיו שנים על שנים במעלה השביעית שהם ד' אלפי אלפים ונשאר לנו המספר הנז' | ||||||||
|
והנה נכפול אלפים במאתים פעמים יהיה ת"ת אלף ונשארו קצ"ט אלפים גם תרצ"ו | ||||||||
|
נשוב לכפול אלפים על ל' פעמים יעלו ק"כ אלף | ||||||||
|
נסירם מהמספר הנשאר ישארו ע"ט אלף וגם תרצ"ו | ||||||||
|
נשוב לכפול אלפים על ו' פעמים[54] יעלו כ"ד אלף | ||||||||
|
נסירם מהמספר הנשאר וישארו נ"ה אלפים גם תרצ"ו | ||||||||
|
וכבר כפלנו האלפים על כל המספרים | ||||||||
|
עתה נחל לכפול מאתים על עצמו ועל האחרים שהם אחריו | ||||||||
|
והנה כפלו על עצמו מ' אלפים | ||||||||
|
חסרנום מהמספר הנשאר ישארו ט"ו אלפים תרצ"ו | ||||||||
|
גם נכפול ר' על ל' פעמ' יעלו י"ב אלף | ||||||||
|
נחסרנו מהמספר הנשאר ישארו ג' אלפים תרצ"ו | ||||||||
|
ועוד נכפול ר' על ו' פעמ' יעלו אלפים ות' | ||||||||
|
נסירם מהמספר הנשאר ישארו אלף ורצ"ו | ||||||||
|
וכבר השלמנו לכפול ר' על כל המספרים שהם אחריו | ||||||||
|
נחל מן ל' | ||||||||
|
והנה כפלו על עצמו תת"ק | ||||||||
|
נחסרנו מהמספר הנשאר ישארו שצ"ו | ||||||||
|
נכפול עוד ל' על ו' פעמים יהיו ש"ס ונשארו ל"ו שהוא מרובע ו' והנה החשבון נכון | ||||||||
| Before I will discuss the numbers that have no root, I shall show you a method how you can know many square numbers from one square number and many roots from one root: | וקודם שאדבר על המספרים שאין להם שרש אראה לך דרך איך תוכל לדעת מרובעים רבים ממרובע אחד גם שרשים רבים משרש אחד | ||||||||
| Know that the product of a square by a square is always a square and its root is the product of the root of one of the squares by the root of the other square.
|
ודע כי כפל מרובע על מרובע לעולם יהיה מרובע והשורש יהיה כמו העולה מכפל שרש אחד מהמרובעים על שרש מרובע האחר | ||||||||
|
דמיון כפלנו מרובע ה' על מרובע ט' ועלה אלפים וכ"ה וזה החשבון מרובע בקשנו לדעת כמה שרשו כפלנו ה' על ט' ועלו מ"ה והוא השרש באמת | ||||||||
| The ratio of a square to a square is also a square. | וערך מרובע אל מרובע גם הוא מרובע | ||||||||
| If you divide the larger one by the smaller one, you will find its root. | ואם חלקת הגדול על הקטן תמצא שרשו | ||||||||
|
דמיון מה ערך מ"ט אל ק' | ||||||||
|
הנה הוא כפלו וב' שביעיות שביעית | ||||||||
|
בקשנו לדעת שרש זה המרובע | ||||||||
|
חלקנו י' על ז' עלה אחד וג' שביעיות וזהו השרש | ||||||||
|
כי אחד על אחד אחד | ||||||||
|
וכפל אחד על ג' שביעיות פעמי' ו' שביעיות | ||||||||
|
וכפל ג' שביעיות על ג' שביעיות ט' שביעיות שביעית | ||||||||
|
והנה נעשה מן הז' שביעיות שביעית אחת ונחברנה אל הו' שביעיות שהיו לנו יעלו אחד שלם | ||||||||
|
והנה יש לנו שנים שלמים וב' שביעיות שביעית | ||||||||
| We wish to know the square of a known number from a known square of a known number: we divide the greater known number by the smaller known number, whose square we know; we take the square of [the quotient] and multiply it by the known square; the result is the square of the greater number that we knew.
|
בקשנו לדעת מרובע מספר ידוע ממרובע ידוע למספר ידוע חלקנו המספר הידוע הגדול על מספר הידוע הקטן שידענו מרובעו ונקח מרובעו ונכפלנו על המרובע הידוע והעולה הוא מרובע המספר הגדול שידענו | ||||||||
|
דמיון בקשנו לדעת מרובע י"ט ממרובע ז' שהוא מ"ט | ||||||||
|
חלקנו י"ט על ז' עלו ב' וה' שביעיות | ||||||||
|
כפלנו מרובע זה והנה ב' על ב' ד' שלמי' | ||||||||
|
וב' על ה' שביעיות פעמי' הם כ' שביעיו' | ||||||||
|
וה' על ה' הם כ"ה שביעיות שביעית | ||||||||
|
נעשה מהם ג' שביעיות נשאר לנו ד' שביעיות שביעית נחבר הג' שביעיות שהיו לנו על הכ' יהיו כ"ג | ||||||||
|
והנה המרובע ז' שלמים וב' שביעיות וד' שביעיות שביעית | ||||||||
|
והנה נכפול מ"ט על ז' יעלו שמ"ג נחבר אליהם י"ד שהם ב' שביעיות מ"ט יהיו שנ"ז | ||||||||
| If we sum 2 squares, whether they are successive or far apart from each other, we double the sum, then subtract from this double the square of the difference between their two roots. The remainder is always a square, and the sum of the two roots is [its] root. | ואם חברנו ב' מרובעים בין שיהיו על הסדר או שיהיו מרוחקי' זה מזה נכפול המחובר ונחסר מזה הכפל מרובע היתרון שיש בין שני המספרי' שהם השרשים יהיה הנשאר לעולם מרובע והמחובר מהב' שרשים הוא השרש | ||||||||
|
דמיון חברנו פ"א שהוא מרובע ט' עם תשכ"ט שהוא מרובע כ"ז יהיו תת"י וכפלו אלף ותר"כ וידענו כי שרש המרובע הקטן ט' ושרש המרובע הגדול כ"ז והיתרון ביניהם י"ח ומרובעו שכ"ד חסרנום מהכפול נשאר אלף ורצ"ו והוא מרובעו ושרשו ל"ו שהוא מחובר מט' עם כ"ז | ||||||||
| If we sum 3 squares, we triple them, then subtract from the product the [sum of] squares of the 3 differences that are between the roots of the 3 numbers. The remainder is also a square. If you sum the 3 roots, the sum is the root of the remainder. | ואם חברנו ג' מרובעי' ונכפלם ג' פעמים ונחסר מהעולה באחרונה מרובעי הג' יתרונים שיש בין שרשי הג' מספרים והעולה באחרונה יהיה גם הנשאר מרובע וכאשר תחבר הג' שרשים יהיה המחובר שרש הנשאר | ||||||||
|
דמיון חברנו ל"ו שהוא מרובע ו' עם ס"ד שהוא מרובע ח' ועם ד' מאות שהוא מרובע כ' והנה המחובר מג' מרובעי' הוא ת"ק כפלנוהו ג' פעמים עלו אלף ות"ק שמרנו זה החשבון | ||||||||
|
והמחובר מן הג' שרשים הוא ל"ד והוא שרש מרובע הנשאר | ||||||||
| Likewise, if you sum 4 or 5 numbers and multiply them a certain number of times. | ועל זה הדרך אם חברת ד' מספרים או ה' ותכפלם כך פעמ' | ||||||||
| The rule is that you multiply by the number of squares that you sum, then always subtract the squares of the differences, as many as they are, from the sum; the remainder is a square. You just have to be sure to know the differences. | והכלל שתכפול כפי מספר המרובעי' שחברת ותחסר לעולם מרובעי היתרוני' כמה שיהיו מהמחובר יהיה הנשאר מרובע ויש לך להישמר לדעת היתרונים | ||||||||
| I will tell you [a rule] according to which you can know how much the number of differences is: always subtract one from the number of squares and know how much is the sum of all numbers that precede this number, as I showed you and as the sum so is the number of differences. | ואומר לך שתוכל לדעת ממנו כמה מספר היתרונים חסר לעולם אחד ממספר המרובעי' ודע כמה מספר המחובר שלפני אותו המספר כאשר הראיתיך וכמספר המחובר יהיה המספר היתרונים | ||||||||
|
דמיון חברנו ו' מרובעי' ונרצה לדעת מספרי היתרוני' | ||||||||
|
נחסר אחד כאשר דברנו ישארו ה' והמספרי' שהם לפניו יהיו ט"ו וככה יהיו מספרי היתרוני' | ||||||||
Fractions |
|||||||||
| Now we start to discuss the square fractions that have a true root: | עתה נחל לדבר על הנשברי' המרובעי' שיש להם שרש אמת | ||||||||
| We say a general statement about the square fractions that are alone or combined with integers: | ונאמ' דבר כולל לנשברי' המרובעי' שהם לבדם או שהם עם שלמי' | ||||||||
| See if the fraction is one-half, third, fifth, sixth, seventh, or eighth, it is not a square. | הסתכל אם היה הנשבר חצי או שלישית או חמישית או ששית או שביעית או שמינית אינו מרובע | ||||||||
| This is because these numbers as integers are not squares. | והיה כן בעבור כי אלה המספרי' בשלמי' אינם מרובעי' | ||||||||
| Only if there is one-quarter, or one-fifth of one-fifth, one-sixth of one-sixth, one-seventh of one-seventh; or one-eighth of one-eighth, or one-half of one-eighth, for it is one-sixteenth. | רק אם היה בו רביעית או חמישית חמישית או ששית ששית או שביעית שביעית או שמינית שמינית או חצי שמינית כי הוא חלק מי"ו | ||||||||
| Observe: if there is one-quarter in square, know that there is one-half in the root; if there is one-ninth there, know that there is one-third in the root; if there is one-half of one-eighth there, know that there is one-quarter in the root. | והנה הסתכל אם היה במרובע רביעית דע כי בשרש חצי ואם שם תשיעית דע כי יש בשרש שלישית | ||||||||
| We begin to discuss the square fractions that are alone without integers: | ונחל לדבר על המרובעי' נשברי' לבדם שאין עמהם שלמי' | ||||||||
| We have already said that the fractions are reversed to the integers. | וכבר אמרנו כי הנשברי' הפך השלמי' | ||||||||
| When you multiply an integer by and integer whether by itself or by another, the product is greater than the [multiplied] numbers; the reverse is with fractions. | וכאשר תכפול חשבון שלם על חשבון שלם בין יהיה על עצמו או על אחר יהיה העולה גדול מהמספרי' והפך זה בנשברי' | ||||||||
| Also, while the squares of the integers are greater than their roots, the opposite is for the square fractions: their roots are greater than their squares. | וכאשר המרובעי' השלמי' גדולי' משרשיהם יהיו המרובעי' הנשברי' להפך בי' שרשיהם גדולים ממרובעיהם | ||||||||
| Extracting the squares from their roots is easy: for, the product of one-half by one-half is one-quarter and this is the square; likewise one-third by one-third is one-ninth; 2 thirds by 2 thirds are one-third; one-third of one-third is one-ninth; 4 fifths by 4 fifths are 3 fifths and one-fifth of one-fifth and this is the square. | ולהוציא המרובעי' משרשיהם דבר קל כי כפל חצי על חצי הוא רביעית אחד והוא המרובע וככה שלישית על שלישית תשיעית אחת | ||||||||
| In this way for all. | ועל זה הדרך הכל | ||||||||
| But, it is difficult to extract the root from the square. | רק הקשה להוציא השרש מהמרובע | ||||||||
| I shall give you a general way in the way of the astrologers that extract all their numbers from the number 60. | ואתן לך דרך כוללת על דרך חכמי המזלות שיוציאו כל חשבונם מחשבון ס' | ||||||||
|
אם ישאל אדם כמה הם ב' שלישיות כפולות על ב' שלישיות | ||||||||
|
תכפול מ' על מ' יהיו אלף ות"ר חלקם על ס' יהיו כ"ו חלקי' ראשוני' ונשארו מ' שניים והכל ד' תשיעיות | ||||||||
|
| |||||||||
|
כי תשיעית ו' ראשוני' גם מ' שניים שהם שתי שלישיות ראשון ואם עשינו מזה המספר שלישיות יהיו כ' גם נכפול ס' על ג' יהיו ק"פ והכל יהיו ממתכונת אחת וכאשר נחלק זה המספר על כ' עלו ט' שהוא תשיעית אחת | ||||||||
|
ואם הפך השואל השאלה ואמר כי המרובע ד' תשיעיות אחד כמה השרש | ||||||||
|
הפוך גם אתה הדבר שתדע כמה הם ד' תשיעיות ס' וכבר אמרנו שהם כ"ו ראשוני' ומ' שניי' עשה מן הראשוני' שני' ושים השניי' עמהם יהיו הכל אלף ת"ר וזה המספר בזוגות דומה לי"ו וחשוב שהם שלמי' והנה השרש מ' שוב וחשוב כי הם ראשוני' וככה הוא השרש | ||||||||
|
דמיון בחשבון שלא יתחלק על ס' כפלנו ד' שביעיות על ד' שביעיות | ||||||||
|
והוא על דרך חכמי החשבון נכפול ד' על ד' יהיו י"ו | ||||||||
|
ועל דרך הדומה לחכמי המזלות יהיו חלקיו ע' והנה ד' שביעיות הם מ' נכפול מ' על מ' יהיו אלף ות"ר | ||||||||
|
ואם השואל יהפוך השאלה ויאמר כמה שרש זה המרובע שהוא ככה | ||||||||
|
גם אנחנו נהפוך הכ"ב ראשוני' ונעשה מהם שניי' ונוסיף עליהם הס' שניים שהיו לנו יהיו אלף ות"ר נחשוב כי הם שלמי' ושרשם מ' ונחשוב כי הם ראשוני' וזהו השרש באמת | ||||||||
|
ואם רצינו להשיב ממתכונת ע' אל מתכונת ס' | ||||||||
|
נכפול מ' על ס' ונחלק העולה על ע' יעלו ל"ד וישארו כ' נכפלם פעם אחרת על ס' ונחלק העולה על ע' יעלו י"ז וישאר לנו זה שאמר שהוא אחד הוא עשרה לכן החשבון אינו מדקדק נעשה ממנו ס' ובעבור כי ע' גדול מס' נכפלנו עוד ויהיו ג' אלפים ות"ר | ||||||||
|
דמיון אחר כפלנו שלישית על שלישית | ||||||||
|
עלה תשיעית אחד והוא המרובע ונעשה חלקיו צ' ושלישיתו ל' | ||||||||
|
והפך זה בקשנו לדעת השרש מזה המרובע | ||||||||
|
עשינו מהי' חלקים שניים והם תת"ק חשבנו שהם שלמי' וכמספר הזה יהיו ראשוני' והוא השרש | ||||||||
| All of this is true if there is a real square number and a real root, but not if it is said about fractions that have no real root, whether divisible by 60, or not. | וכל זה נכון אם יש מרובע אמת ושרש אמת רק אם אמר חשבון חלקים שאין להם שרש אמת בין שיהיה להם ערך אל ס' או לא יהיה | ||||||||
|
כי אם אמר י"ב חלקים הוא המרובע כמה השרש | ||||||||
|
ידענו כי י"ב הוא חמישית ס' וכבר אמרנו כי לא יתכן להיות במרובע חמישית | ||||||||
|
וככה אם אמר כי המרובע י' חלקים שהוא ששית אחת לא יתכן להיות | ||||||||
|
רק אם אמר כי המרובע חלק אחד ומ' שניים שהוא ששית הששית הוא הנכון | ||||||||
| As for the numbers that are divisible by 60, most of them are not squares. | והנה במספר שיש לו ערך אל ס' לא יתכן להיות רובי המספרים מרובעים | ||||||||
| All the more so the numbers that are not divisible at all, such as 11, 13, (15), 17, 19 and many others that have no divisor. | אף כי המספרים שאין להם ערך כלל כמו י"א י"ג ט"ו י"ז י"ט ואחרים רבים שאין להם ערך | ||||||||
| [Latter], I will give you a method by which you will be able to extract for every square fraction its root by approximation to the truth. | ועוד אתן לך דרך שתוכל להוציא לכל מרבע נשבר שרשו בדרך שהיא קרובה אל האמת | ||||||||
Integers and Fractions |
|||||||||
| Now, I shall discuss the integers with fractions that are squares. | ועתה אדבר על השלמים שיש בהם נשברים והם מרובעים | ||||||||
|
דמיון אמר אומר מרובע שהוא י"א ותשיעית כמה השרש | ||||||||
|
אחר שאמר שיש בו תשיעית נכון הוא להיותו מרובע ובשרשו שלישית נכח התשיעית חסר ממנו התשיעית שהוא מרובע שבר השבר נשארו י"א שלמים | ||||||||
|
דמיון אחר המרובע שהוא ז' שלמים ונ' חלקים ראשונים וכ"ד שניים | ||||||||
|
הנה בעבור ששם כ"ד שניים ידענו שיש בחשבון חומש החומש שהוא ב' חלקים וכ"ד שניים נחסר זה מרובע השבר מהמספר הנתון ישאר ז' שלמים ומ"ח ראשונים והנה המרחק מהמרובע שהוא אחריו אחד שלם וי"ב חלקים שהם ע"ב ראשונים | ||||||||
|
והנה נבחן זה בכפל כי הנה ב' על ב' ד' שלמים וידענו כי ב' על מ"ח פעמים יהיו קצ"ב ומ"ח הם ד' חמישיות יהיו י"ו חמישיות נעשה מהם ג' שלמים וישאר חמישית אחת | ||||||||
|
דמיון מרובע שהוא מ"ד וד' תשיעיות | ||||||||
|
ידענו כי התשיעיות מן השלישיות יצאו | ||||||||
|
והנה נסיר זה המרובע שהוא לשברי שברים ונבקש כמה מרחק השלמים מן המרובע שעבר והנה ח' נשיבם ראשונים יהיו ת"פ | ||||||||
|
ובדרך חכמי המזלות נכפול ו' על ו' והם ל"ו ונכפול ו' על מ' ומ' על ו' והם ת"פ ראשונים נחלקם על ס' ועלו ח' שלמים | ||||||||
| After I have mentioned these examples, proceed in this way with all the ranks. | ואחר שהזכרתי אלה הדמיונים תעשה כדרך הזה בכל המעלות | ||||||||
Approximations |
|||||||||
| Now I will tell you a general way for all the numbers that have a root or do not have [a root]: | ועתה אומר לך דרך כלל לכל המספרים שיש להם שרש אמת או אין להם | ||||||||
| Know that between two successive [square] numbers there are always [numbers] as the number of the roots of both. | דע כי לעולם יהיה בין ב' מספרים שהם על הסדר כמספר השנים שרשים | ||||||||
| Look at the number you want: how much is its distance from the preceding square? If the difference between your number and the preceding square is as the root of the preceding square, it is the mean number.
|
והנה הסתכל במספר שתרצה כמה מרחקו מהמרבע שעבר אם היה המרחק בין מספרך ובין המרובע שעבר כשרש המרובע שעבר הוא מספר האמצעי | ||||||||
| Extract any number that is less than the mean from the preceding square number.
|
וכל מספר שיהיה פחות מהאמצעי הוציאהו מהמספר המרובע שעבר | ||||||||
| If it is greater than the root of the preceding [square], extract it backwards from the succeeding square.
|
ואם היה יותר מהשרש שעבר הוציאהו אחורנית מהמרובע שלפניו | ||||||||
|
דמיון המספר עשרים | ||||||||
|
והנה מרחקו מן המרובע שעבר ד' ואם תשיבם ראשונים ותחלקם על ח' שהוא כפל השרש יהיו ל' שהוא חצי ס' | ||||||||
|
ואם לקחנו מרחק הכ' מהמרובע הבא יהיה כמספר השרש נשיבם ראשונים ונחלקם על י' שהוא כפל השרש יהיו ל' | ||||||||
|
על כן אמרתי כי כ' הוא חשבון אמצעי | ||||||||
| Now, note: if your number is close to the preceding square, look at the difference between them, convert it to primes. | ועתה שים לבך אם היה מספרך קרוב אל המרובע שעבר ראה המרחק שיש ביניהם ועשה ממנו ראשונים | ||||||||
| If there are primes in your number, add them to the primes you got. | ואם יש בחשבונך ראשונים הוסיפם על הראשונים שעשית | ||||||||
| If you also have seconds, convert all to seconds. | ואם יש עמך שניים השב הכל במערכת השניים | ||||||||
| Or choose the following shorter way: if the seconds are less than 30, leave them out; if they are more, add one prime instead of them. | או קח דרך קצרה אם היו השנים פחותים מל' הניחם ואם יותר הוסף ראשון אחד עליהם | ||||||||
| When you know of how many primes the difference is, divide it by twice the preceding root and add the quotient to the preceding root. The sum is called "the first root". | וכאשר תדע כמה הראשונים של המרחק חלקם על כפל השרש שעבר וההוה הוסיפנו על השרש שעבר והמחובר יקרא שרש ראשון | ||||||||
| If your number is in hundreds or thousands, this root is enough for you in geometry, because it does no harm. | ואם חשבונך היה במאות ואלפים זה השרש יספיק לך במדות כי לא יזיק | ||||||||
| However, if the number is small, you still need a second root, which is more precise. | רק אם המספר היה קטן אתה צריך לשרש שני שהוא יותר מדוייק | ||||||||
| To calculate chords and arches, you need a third root, which is even more precise. | ולהוציא היתרים והקשתות אתה צריך לשרש שלישי שמדוייק יותר | ||||||||
| This is how the approximation is applied: when you have the primes of the difference, know how much is their square, divide it by twice the first root, then know how much the quotient is, and in what rank it is, whether in primes or in seconds, as I have shown you in the chapter of the fractions and subtract it from the first root; the remainder is the second root. | וככה יהיה הדקדוק כשיהיו לך הראשונים של המרחק דע כמה מרובעם וחלקהו על כפל השרש הראשון ודע העולה כמה הם ובאיזה מעלה הם בראשונים או בשניים כאשר הראיתיך בשער השברים וההוה חסרהו מן השרש הראשון והנשאר הוא השרש השני | ||||||||
| If you want to calculate it more precisely, take the square of the quotient and divide it by double the second root. | ואם תרצה לדקדקו עוד קח מרובע זה שעלה בחלוק וחלקהו על כפל השרש השני | ||||||||
| Now I shall give you examples according to way of the arithmeticians with approximate calculation. | ועתה אתן לך דמיון על דרך חכמי החשבון בדרך קרוב | ||||||||
|
בקשנו לדעת שרש מאתים | ||||||||
|
והנה המרובע שעבר קצ"ו והמרחק ד' שלמים כשתחלקם על כ"ח שהוא כפל השרש תעלה שביעית אחת והנה השרש י"ד ושביעית אחת | ||||||||
|
| |||||||||
|
ואם רצית לדעת שרש עשרים אלף | ||||||||
|
כפול זה השרש על י' יעלה קמ"א וג' שביעיות | ||||||||
|
ואם בקשנו לדעת כמה שרש שנים משרש מאתים שהוא י"ד ושביעית | ||||||||
|
נקח עשיריתו והנה מי' נקח אחד שלם ועשירית ד' והם ד' עשיריות וידענו כי ד' עשיריות הם ב' חמישיות שהם כ"ד ראשונים | ||||||||
|
| |||||||||
|
נשוב להוציא זה השרש מכ' אלף | ||||||||
|
וידענו כי זה החשבון הוא דומה לאחדים וי' אלפים כמו אחד והוא המרובע הנמשל | ||||||||
|
והנה נחסר י' הפירוש שתקח הראשונים של המרחק אחר שלקחת אותם על כפל השרש שעבר והם הראשונים אשר עלו לך בשרש | ||||||||
| Or if you wish, say that you take the first root, square it and see how close it is. Then, divide its excess over your first number by double the first root, subtract the quotient from the first root and the remainder is the second root. | או אם תרצה אמור שתקח השרש הראשון ותרבעהו ותראה כמה יהיה הקרוב והתוספת אשר בו על מספרך הראשון והתוספת ההוא תחלק על כפל השרש הראשון והיוצא בחלוקה תחסר מהשרש הראשון והנשאר הוא השורש השני | ||||||||
|
ת"ו אלפים ממספרנו ישארו י' אלפים נחלקם על כפל השרש שהוא ר' ולא נתן לו כל מה שנוכל אך נניח כפי מרובע החלוק והנה נתן לו מ' וישארו לנו אלפים נסיר מהם אלף ות"ר שהוא מרובע החלוק נשאר ת' והשרש שלנו ק"מ וכפלו ר"פ נחלק הנשאר עליו נתן לו אחד נשארו ק"כ נחסר ממנו א' שהוא מרובע א' נשאר קי"ט והשרש שלנו קמ"א נעשה מהנשארים ראשונים יהיו ז' אלף וק"מ נחלקם על רפ"ב שהוא כפל השרש שלנו עלו כ"ה חלקים ראשונים וי"ט שניים נחלק כל מה שאמרנו מן השלמים והשניים על ק' יהיה העולה אחד שלם כ"ד נ"א י"א וזהו מדוקדק יותר מן הראשון שהזכרנו | ||||||||
| If you take any number that is twice a square number and multiply the root of this square number by this root, you will get the root of the number exactly. | ואם תקח כל חשבון שהוא כפל מרובע ותכפול שרש המרובע על זה השרש יצא לך שרש החשבון מדוקדק | ||||||||
|
דמיון רצינו לדעת כמה שרש י"ח | ||||||||
|
והנה כפלנו שרש המרובע שזה המספר כפלו ועלה ד' שלמים י"ד ראשונים ל"ג שניים ל"ג שלישיים | ||||||||
|
| |||||||||
|
ואם כפלנו זה המספר יהיה שרש ע"ב שהוא כפל כפל י"ח | ||||||||
|
ואם לקחנו חצי זה שרש יהיה שרש ד' וחצי שהוא רביעית י"ח | ||||||||
|
ואם נקח מרובע ז' אלפים ור' שהוא כפל מרובע ס' יהיה השרש פ"ד שלמים נ"א ראשונים י"א שניים וזהו שרש שנים בעצמו כי השיבונום בדרך ראשונים והנה חשוב אלה פ"ד שהיו שלמים חשבם ראשונים והראשונים שניים והשניים שלישיים | ||||||||
|
| |||||||||
|
ואם תכפול זה המספר על עצמו אחר שתשיבם שלישיים ותחלקם במשפט שתשיבם אל המעלה הראשונה לא ישאר לך שני אחד ואף כי ראשון | ||||||||
|
נשוב להוציא שרש שנים להיותו דמיון לאחדים הנה המרובע שעבר הוא אחד והמרחק בין חשבוננו ובינו הוא אחד נשיבנו ראשונים יהיו ס' נחלקם על כפל השרש שהוא שנים יהיו ל' ראשונים והנה השרש הראשון א' ול' ראשונים | ||||||||
|
| |||||||||
|
ואינו נכון בעבור שהוא בחשבון קטן כי הנה כאשר הוציאנו אותו מחשבון ר' היה קרוב אל האמת ומהחשבון כ' אלף יותר מדוקדק ויספיק לנו השרש הראשון | ||||||||
|
והנה עלה לנו בחלוק ל' חלקים ראשונים ומרובעו ט"ו ראשונים כי כפל ראשונים על ראשונים שניים והם ט' מאות נחלקם על ס' יהיו ט"ו ראשונים נחלקם על כפל השרש שהיה לנו הוא ג' יעלו ה' | ||||||||
|
| |||||||||
|
נשוב ונקח מרובע החלוק שהוא כ"ה והם שניים והשרש השני שהיה לנו שהיה אחד שלם וכ"ה ראשונים יהיה כפלו ב' שלמים נ' ראשונים נחלק השניים על זה ובעבור שיש לנו ה' ששיות נשיב הכל מערך ו' והנה נכפול כ"ה על ו' יהיו ק"נ נחלקם על י"ז נתן לו ח' ונשארו י"ד נשיבם ממערכת ס' יהיו תת"מ נחלקם על י"ז עלו מ"ט והם שלישיים ונשארו ז' מי"ז נשליכם כי אין צורך אליהם כי יש לנו עוד לחסר מרובע שעלה בחלוק עתה והנה כאשר נחסר ח' שניים גם מ"ט שלישיים מהשרש השני יהיה הנשאר אחד שלם כ"ד ראשונים נ"א שניים י"א שלישיים | ||||||||
|
| |||||||||
|
ואילו עשינו על דרך חכמי המזלות יהיה הדבר שוה | ||||||||
|
ואם היינו מדקדקים עוד מדרך מרובע ח' שניים מ"ט שלישיים שאמרנו היה יוצא השרש מדוייק שאין דיוק כמוהו א' כ"ד ראשונים נ"א שניים י"ז שלישיים נ"ד רביעיים | ||||||||
|
| |||||||||
|
בקשנו להוציא שרש י' | ||||||||
|
והנה המרחק מהמרובע שעבר אחד נשיבנו ראשונים יהיו ס' | ||||||||
|
| |||||||||
|
נשוב לדקדקו והנה נקח הק' שהוא מרובע החלוק ונחלקנו על ו' ושלישית שהוא כפל השרש הראשון ונשיב הכל מערך ג' | ||||||||
|
| |||||||||
|
ואם נדקדקנו יותר יהיה ג' שלמים ט' ראשונים מ"ד שניים י"ב שלישיים | ||||||||
|
וכשנכפול זה החשבון על י' יהיה העולה ל"א שלמים ל"ז ראשונים כ"ב שניים וזהו שרש אלף | ||||||||
|
ואם נכפלנו על אלף נמצא שרש עשרת אלפי אלפים | ||||||||
|
שאלה חלקנו שרש י"ח על שרש ח' כמה יעלה בחלוק | ||||||||
|
ידענו כי אין לי"ח שרש ג"כ לח' והנה נעשה דרך אחרת שנחלק י"ח על ח' ויעלה בחלוק ב' ורביע וזה החשבון מרובע ושרשו אחד וחצי והנה כאשר נוסיף על כפל שרש ב' חציו תמצא שרש י"ח מדוקדק | ||||||||
|
שאלה הצבנו סולם אל קיר י' אמות גבהותו וככה גבהות הסולם הורדנו ראש הסולם מלמעלה ב' אמות נבקש לדעת כמה יהיה מרחק הסולם מיסוד הקיר | ||||||||
| I will give you a rule concerning this matter: the square of the remainder from the top of the ladder plus the square of the distance of the foot from the base are always equal to the square of the ladder. | אתן לך כלל בדבר זה לעולם יהיה מרובע הנשאר מראש הסולם עם מרובע מרחק הרגל מן היסוד שוים אל מרובע הסולם | ||||||||
|
והנה חסרנו ב' אמות שירד הראש מתחלת הקיר נשארו ח' ומרובעו ס"ד נחסרנו מק' שהוא מרובע הסולם ישארו ל"ו ושרשו ו' וככה הוא מרחק הסולם למטה מן היסוד | ||||||||
|
| |||||||||
|
דמיון אחר הורדנו הראש אמה כמה המרחק מן היסוד | ||||||||
|
חסרנו א' מי' ונשארו ט' ומרובעו פ"א נחסרנו מק' ישארו י"ט וזהו מרובע המרחק וככה נוציא שרשו | ||||||||
The Circle |
|||||||||
| Now we shall start talking about the circle as it depends on the root: | ועתה נחל לדבר בעגול יען שהוא תלוי בשרש | ||||||||
| Know that there are many matters in the circle: one is the perimeter, the second is the diameter, the third is the multiplier [= pi], the fourth is the chord, the fifth is the versed sine, the sixth is the area. | דע כי יש בעגול דברים רבים האחד קו העגול והשני האלכסון והשלישי הכפל והרביעי היתר והחמישי החץ והששי השברים | ||||||||
| You can extract each of them that is unknown from two that are known and there are those among them of which one that is unknown can be known from another, as I will explain and give an example for each. | ותוכל להוציא אחד מהם שאינו ידוע מב' שהם ידועים ויש מהם שיוכל לדעת אחד מהם שאינו ידוע מאחר כאשר אפרש ואתן דמיון לכל אחד ואחד | ||||||||
|
נחשוב עגול אלכסונו י' וחצי היתר ד' והחץ ב' כמה האלכסון | ||||||||
|
חלקנו מרובע חצי היתר על החץ והוספנו החץ בעצמו על מה שעלה בחלוק והמחובר הוא האלכסון | ||||||||
|
דמיון אחר באלכסון י' והחץ ג' ומרובע חצי היתר כ"א | ||||||||
|
חלקנוהו על החץ עלה ז' והוספנו עליו ג' והנה הוא האלכסון | ||||||||
| A way to extract the chord from the versed sine and the diameter: | דרך להוציא היתר מן החץ ומן האלכסון | ||||||||
|
הנה חצי האלכסון ה' והחץ אחד נחסרנו מה' ישאר ד' אל הנקודה ומרובעו י"ו | ||||||||
|
| |||||||||
|
והנה כל היתר ו' | ||||||||
| This matter should be a foundation for you, as the square of what remains from the versed sine to the midpoint plus the square of half the chord are equal the the square of half the diameter. | וזה הדבר יהיה לך יסוד כי לעולם מרובע מה שנשאר מן החץ אל הנקודה עם מרובע חצי היתר יהיו שוים אל מרובע חצי האלכסון | ||||||||
| Another way to extract the chord: multiply the versed sine by the remainder from the diameter; the product is the square of half the chord. Extract its root and double it; then you will find the whole chord. | דרך אחרת להוציא היתר כפול החץ על כל הנשאר מן האלכסון והעולה הוא מרובע חצי היתר קח שרשו וכפלהו ותמצא כל היתר | ||||||||
| Another method: See how much the ratio of the versed sine to the whole diameter is; take the same from the square of the diameter and this is the sum of the square of the versed sine and the square of half the chord. The ratio of the square of the versed sine to the [sum of] the square of the versed sine and the square of half the chord is as the ratio of the versed sine to the entire diameter. | דרך אחרת ראה כמה ערך החץ אל כל האלכסון וככה תקח ממרובע האלכסון והוא המחובר ממרובע החץ וממרובע חצי היתר וערך מרובע החץ אל מרובע החץ ומרובע חצי היתר כערך החץ אל כל האלכסון | ||||||||
|
דמיון בעגול הנזכר החץ ב' והנה ערכו אל כל האלכסון החמישית והנה חמישית ק' שהוא מרובע כל האלכסון כ' וזה המספר כולל מרובע החץ ומרובע חצי היתר וראוי להיות מרובע החץ חמישית כ' שהוא ד' נחסרם מהכ' ישארו י"ו והוא מרובע חצי היתר | ||||||||
|
דמיון אחר בחשבון שיש לו חלקים ונאמר כי החץ ג' שלמים וכ' חלקים | ||||||||
|
וזה ערכו אל י' שלישית הנה נקח שלישית ק' שהוא מרובע האלכסון והוא ל"ג שלמים וו' ראשונים ומ' חלקים שניים והוא מרובע החץ נחסרם מל"ג וישארו כ"ב י"ג כ' והוא מרובע חצי היתר | ||||||||
| I shall give you a general way for the concepts of circle: | ואתן לך דרך כלל בדברי העגול | ||||||||
| We know that 2 equal diameters are cutting the circle. | ידענו כי ב' אלכסונים שוים מחלקים העגול | ||||||||
| If the versed sine is one-third of the diameter, the square of half the chord is its double; together they are three [times]. | ואם החץ שלישית האלכסון יהיה מרובע חצי היתר כפלו בין שניהם הם ג' | ||||||||
| If the versed sine is one-quarter of the diameter, the square of half the chord is triple the square of the versed sine. | ואם החץ רביעית האלכסון יהיה מרובע חצי היתר ג' כפל מרובע החץ | ||||||||
| In this way for every number. | ועל זה הדרך כל החשבון | ||||||||
|
שאלה אם אמר החץ כ' חלקים | ||||||||
|
זה הערך תוכל לקחתו על שני דרכים | ||||||||
|
האחד שתשיב האלכסון כלו ראשונים יהיו ת"ר ותעשה הערך כך | ||||||||
|
| ||||||||
|
והנה נכפול ב' בק' מרובע האלכסון יהיו אלפים נחלקם על ת"ר יהיו ג' שלמים ושלישית אחד שהם ב' חלקים | ||||||||
|
ואם שבנו עוד להפריד מרובע החץ ממרובע חצי היתר נקח מג' כ' ערך ג' כ' אל ק' והיה ו' מ' והוא מרובע החץ | ||||||||
|
וישאר מרובע היתר ג' י"ג ב' | ||||||||
|
ובדרך אחרת ידענו כי כ' חלקים מי' שלמים הוא שליש העשירית נקח מק' שהוא מרובע האלכסון שליש העשירית יהיה ג' שלמים וכ' חלקים | ||||||||
| A way to extract the versed sine from the chord: | דרך להוציא החץ מהיתר | ||||||||
| Subtract the square of half the chord from the square of half the diameter, take the root of the remainder and subtract it from half the diameter; the remainder is the versed sine. | גרע מרבע חצי היתר ממרובע חצי האלכסון וקח שרש הנשאר וגרעהו מחצי האלכסון והנשאר הוא החץ | ||||||||
|
דמיון אמר כי היתר ו' | ||||||||
|
נקח מרובע חציו שהוא ט' נחסרנו מכ"ה שהוא מרובע חצי האלכסון וישארו י"ו ושרשו ד' | ||||||||
| To extract the perimeter from the diameter | להוציא קו העגול מן קו האלכסון | ||||||||
|
חכמי המדות אמרו כי הקו הסובב הוא ג' מהאלכסון ויותר שביעית והנה הוא בחשבון ז' אל כ"ב | ||||||||
|
ואם כפלת האלכסון על ג' ושביעית יהיה העולה הקו הסובב | ||||||||
|
או אם תכפול האלכסון שתרצה על כ"ב ותחלק העולה על ז' תמצא הקו הסובב | ||||||||
|
והפך זה אם ידעת הקו הסובב ותרצה לדעת האלכסון | ||||||||
|
כפול הקו על ז' וחלק העולה על כ"ב תמצא האלכסון | ||||||||
|
והנה על דעת אלה אם היה האלכסון אחד יהיה הקו הסובב ג' שלמים ח' הראשונים ל"ד שניים י"ז שלישיים | ||||||||
|
וארישמדס החכם נתן ראיה כי הוא פחות מזה המספר כי אמר שהנוסף פחות מי' חלקים מע' | ||||||||
|
גם הביא ראיה כי הנוסף יותר מי' חלקים מע' וחצי והנה הנוסף על השלשה שלמים ח' ראשונים כ"ד שניים ל"ה שלישיים | ||||||||
|
ונתן ראיה כי ראוי להיות יותר מזה המספר | ||||||||
|
ותלמי עשה חשבונו אמצעי כי התוספת ח' ראשונים ל' שניים | ||||||||
|
וחכמי הודו אמרו כי אם היה כ' אלף יהיה הקו הסובב ס"ב אלף ותתל"ח | ||||||||
| When you look closely, you will find it closer than Ptolemy's saying; between them only 3 thirds. | וכאשר תסתכל זה תמצאנו קרוב מדברי תלמי ואין ביניהם כי אם ג' שלישיים | ||||||||
| Since 10 is similar to one and the circle is surrounded by one line, if we set the diameter as 10, the square of the chord is as one-third of the diameter by the perimeter, no more nor less. | ובעבור כי י' דומה לאחד והעגול יסובבהו קו אחד הנה אם שמנו האלכסון י' יהיה מרובע היתר כשלישית האלכסון במספר הקו בלי תוספת ומגרעת | ||||||||
| Likewise, if you make the square between the upper third and the lower third, its area is as the perimeter. | וככה אם עשית מרובע בשלישית העליונה ובשלישית השפלה יהיו שבריו במספר הקו | ||||||||
| We can only know the square of it, which is 987, 5 ninths and 4 eighths of one-ninth that are 53 parts of 81. | רק המרובע נוכל לדעת שהוא תת"ק פ"ז וה' תשיעיות וד' שמיניות תשיעית שהם נ"ג חלקים מן פ"א | ||||||||
| If we set the diameter as 10, we extract the chord from its one-third and construct an [isosceles] triangle on it, so that the area of the triangle is as to the perimeter. | והנה אם שמנו האלכסון י' ונוציא יתר בשלישית ונעשה עליו משולש יהיה רבוע המשלש כקו הסובב | ||||||||
| For every number preceding 10, the ratio of the triangle on the third [of the diameter] to the perimeter is as the ratio [of the number] to 10. | וכל מספר שהוא לפני י' יהיה ערך המשלש בשלישית אל הקו המקיף בערכו אל י' | ||||||||
| If it is greater than 10, the ratio of the perimeter to the triangle on the third is as the ratio of 10 to the diameter. | ואם הוא יותר מעשרה יהיה ערך הקו המקיף אל המשלש בשלישית כערך י' אל הקו | ||||||||
| When we look for the perimeter - how much is it if the diameter is one: | וכאשר נחפש הקו הסובב כמה יהיה אם היה האלכסון אחד | ||||||||
| The perimeter is 3 integers, 8 primes, 33 seconds, 42 thirds and 30 fourths. | יהיה הקו הסובב ג' שלמים ח' ראשונים ל"ג שניים מ"ב שלישים גם ל' רביעיים | ||||||||
| Therefore, the area of the circle, whose diameter is 15, is the root of 5 thousand, no more nor less. | על כן שברי עגול שאלכסונו ט"ו שרש חמשת אלפים בלי תוספת ומגרעת | ||||||||
| In geometry and astrology there is no need to make it accurate. | ובמדות ובחכמת המזלות אין צריך לדקדק זה | ||||||||
|
והנה הוא כאשר אמר ארישמדס שהוא יותר מי' חלקים מע' וחצי וקרוב מאד לדברי חכמי הודו שאין ביניהם רק דבר שאין בו ממש | ||||||||
|
ולדעת הקשתות והמיתרים על דעת חכמי המזלות | ||||||||
|
אדבר עליהם בספר טעמי הלוחות | ||||||||
|
כי הם מבקשים למוד הקו הסובב מהיתרים | ||||||||
|
וחכמי המדות מבקשים לדעת כמה שברים יכילו בעגול | ||||||||
|
ולפי דעתם אם ידעת האלכסון כפול מרובעו על י"א וחלק העולה על י"ד אז תמצא שברי העגול | ||||||||
|
והפך זה אם ידעת כמה שברי העגול ותרצה לדעת כמה האלכסון | ||||||||
| Multiply the area by 14 and divide the product by 11; the quotient is the square of the diameter and its root is the diameter. | כפול השברים על י"ד וחלק העולה על י"א והעולה בחלוק הוא מרובע האלכסון ושרשו הוא האלכסון | ||||||||
| Now I shall talk again about why the arithmeticians subtract one for the foundation. | ועתה אשוב לדבר למה יחסרו חכמי החשבון אחד ליסוד | ||||||||
| Know that one to 9 are the real numbers that correspond to the 9 spheres and all numbers that follow them are similar to them. The similar numbers should be given their ranks from them: 10 in first rank, 100 in the second rank, one-thousand in the third, 10 thousand in the fourth, 100 thousands in the fifth and one-thousand of thousands in the sixth, and so on endlessly. | דע כי אחד עד ט' הם המספרים באמת שהם כנגד ט' עגולות וכל המספרים אחריהם הם נמשלים להם והנה הנמשלים הם ראויים לקחת המעלות מהם והי' ראוים אל מעלה ראשונה והק' בשנית והאלף בשלישית והי' אלף ברביעית וק' אלף בחמישית ואלף אלפים בששית וככה עד אין קץ | ||||||||
| The arithmeticians set the first numbers in the ranks, therefore they had to subtract one to the foundation and this is clear. | וחכמי החשבון שמו המספרים הראשונים במעלות על כן הוצרכו לגרוע אחד ליסוד וברור זה | ||||||||
|
ותראה בדמיון בקשנו לכפול ר' על ש' | ||||||||
|
והנה הנמשלים ב' וג' כפלנו זה על זה והיו ו' והמעלות גם כן הם ו' | ||||||||
| I have already mentioned that in the true calculation the beginning of the fourth rank is 10 thousand and this is the same thing. | וכבר הזכרתי כי בחשבון האמת תחלת הארבעה לי' אלפים הוא והנה הדבר שוה | ||||||||
| They only did so to make it easier for the students. | אך עשו כן כדי להקל על התלמידים | ||||||||
| תם ונשלם תהלה לאל עולם סלה | |||||||||
ראה ספר כליל שפר יסוד מספר שמו נקרא לאברהם בנו מאיר ספרדי אבן עזרא
Additional Excerpt
F14649 ל"ז1
| שאלה ג' אנשים רצו לקנות דג במחיר י"ז פשיטים אמר אחד מהם אני אתן כל מה שיש בידי ואתם תנו חצי מה שיש בידכם | |
| תשובה נבקש מאמר שנוכל להוסיף עליו עד שיהיה המספר ההוא אחר התוספת חציו ושני שלישיותיו ושלש רביעיותיו והוא המורה ובעבור כי מחיר הדג י"ז פשוטים והנה יותר מי"ב | |
| והנה המספר המבוקש פחות מי"ב וכאשר חברנוהו י"ב והוא המורה עם מחיר הדג שהם י"ז יעלו כ"ט | |
| תסיר מהם הי"ז ישארו י"ב נמצאו ביד שנים מהם י"ב פשוטים | |
| וכאשר נוסיף על י"ב חצי הכפל הנשאר שהם ה' מי"ב ועד י"ז וכפלם כ"ה וחציו י"ב וחצי יהיו כ"ד וחצי ויש לך עוד כ"ט ה' וככה ממון ראשונים | |
| וכאשר הוספת על י"ב ו' שיהיה י"ב שתי שלישיותיו היו י"ח הנה יש להשלים עד כ"ט י"ח והוא הממון השני | |
| וכאשר הוספנו על י"ב ד' בלבד עד שיהיו י"ב ג' רביעיותיו והנה היו י"ו וממנו עד כ"ט י"ג והוא הממון השלישי | |
| דבר אחר ממה שיצטרך ידיעתו בחכמה הזאת הוא שהבדל גדול יש בין אמרנו ערך מספר קטן אל הגדול ובין אמרנו ערך מספר גדול אל הקטן | |
| והוא המוזכר אחרון בערך יהיה הראשון הנזכר חלק ממנו ולא יתהפך זה עד שיהיה האחרון חלק מן הראשון וזה יתברר בשני מספרים שאין חלקיהם שוים | |
| המשל בזה כי כאשר אמרנו ערך ג' אל ה' הרצון בו שג' הוא שלשה חלקי הה' ר"ל ג' חמישיותיו | |
| והפך זה אמרנו ערך ה' אל ג' הרצון בו שהחמשה יש בו פעם אחת ג' ושני שלישיותיו | |
| וככה ערך י' אל י"ו הכונה בו שהוא חמש שמיניות של י"ו אבל אמרנו ערך י"ו אל י' הכונה בו שהוא פעם אחת מספר י' וג' חמישיותיו של י' | |
| דבר אחר דע כי כפל מניין על מניין הוא כפל האורך על הרוחב אם שיהיה מרובע רבוע שא' שארכו כרחבו כאמרנו ד' על ד' הם י"ו וגם שיהיה ארכו יותר מרחבו כאמרנו י"ו שהם אמות אומדות שארכם כרחבם כזה או מרובע ארוך כזה | |
| על כן כפל המספר שלמים על שלמים יוסיפו ובו תדע תשבורת הכל אבל כפל שברים על שברים יהיה היוצא ממין השברים | |
| וכשתכפול השברים על השלמים כאמרנו כפול ד' על חצי הוא כאמרנו כפול ד' על חצאים כלומ' שיהיה ארכו ד' אמות ורחבו חצי אמה כזה | |
| כפול שברים על שברים כאמרנו חצי על חצי ויהיה רביעית כלומ' יהיה חצי אמה אורך וחצי אמה רוחב ושברו הוא רביעית | |
| וכאשר נכפול שלמים על שלמים כאמרנו כפול ד' על ד' יהיה מרובעו י"ו מרובעות וכאשר תכפול ב' על ב' יהיה מרובעו ד' | |
| ככה הענין בשברים על כן שלישית על שלישית יהיה תשיעית וכן כל השאר | |
| אבל החלוק הוא שתחלק האורך על הרחב כי לעולם הגדול נחלק על הקטן כי אם חלקנו הקטן על הגדול אותו יקרא ערך ואינו חילוק כי הוא כאמרנו ערך מספר קטן אל מספר גדול | |
| ועל כן כשנחלק שלמים על שלמים יצא בחלוק שלמים כמו שאמרנו | |
| דמיון חלקנו י"ו על ב' עלה ח' הוא שתשבורת הכל השיבונו על קו שיהיה רחבו ב' אם כן יהיה ארכו ח' | |
| ואם חלקנו שלמים על שברים כאמרנו חלקנו י"ו על חצי אחד הנה יהיה ארכו ל"ב ורחבו חצי אחד | |
| ועל כן נחלקנו שברים על שברים ממינם יהיו שלמים | |
| כאמרנו חלקנו ג' רביעיות על ב' רביעיות הוא שנעשה קו ארכו אחד וחצי ורחבו ב' רביעיות כי מה שהיה תשברתו שלשה ו' רביעיות באורך וברוחב חלקנו על הרחב ושמנו רחבו חצי אמה וארכו אמה וחצי | |
| על כן כשנחלק מעלות על מעלות או על ראשונים או על שניים או על איזה חלקים שנרצה יצא בחלוק מעלות שהשיבונו התשבורת כולו אל האורך וקצרנו ברחב | |
| ואם חלקנו ראשונים על שניים יצא בחלוק מעלות גם כן | |
| כי נשיב הראשונים אל השניים ויהיו דומים וישובו מעלות באורך ושניים ברחב ומה שיהיה ישובו מעלות | |
| ואם תחלק הקטן על הגדול ישוב היוצא אל מין אחד גבוה על הקטן והקטן מן הגדול ומזה תבין כל מיני החלוק | |
| שאלה שלשה אנשים באו לקנות דג שמחירו י"ב פשיטי' ומחצה הראשון יתן כל מה שבידו והשני רביעיות והאחרים רביעיתם או השלישי כל מה שבידו והאחרים חמישיתם נרצה לדעת כמה ביד כל אחד | |
| תשובה שמחיר הדג למעלה מי"ב הנה י"ב הוא מספר מכוון שיהיה לנו אחר התוספת שלישית והוא י"ח ורביעית והוא י"ו וחמישית והוא ט"ו הנה כאשר חברנו י"ב על מחיר הדג יהיה כ"ד פשוטים ומחצה | |
| הנה מי"ח עד כ"ד ומחצה הוא ו' מחצה וככה ממון הראשון | |
| ומי"ו עד כ"ד ומחצה ח' ומחצה והוא ממון השני | |
| ומט"ו עד כ"ד ומחצה ט' ומחצה והוא ממון השלישי | |
| ולהוציא אל השאלות והדומות אליהן דע כי חלקי האנשים הן כפי חלקי המורה מחובר עם המחיר | |
| וכאשר רצינו לחדש שאלה דומה לזו הוא שיקח המורה מחובר עם המחיר | |
| ולכן כשנרצה להוסיף עליהם החלקים שנוכל להוסיף עליו ונחבר כל החלקים עמו ונראה כמה עולה עם החלקים וגם החלקים לבד נקח כל העולה והעולה הוא מחיר הדג או מחיר דבר קנוי | |
| דמיון לחלקים כשהם ב' אנשים לבד | |
| נקח ד' על דרך משל המורה וכאשר נכפלהו היו ח' וכאשר נוסיף על ד' שלישית מלבד יהיו ו' הנה הוספנו על זה האחרון ב' על ד' | |
| וכאשר נחבר ב' על ח' עלו י' והוא מחיר הדג | |
| והנה המורה ד' כמו שאמרנו נחברהו על המחיר שהוא י' ויהיו י"ד והנה הראשון שכפל המורה והיו ח' הנה מח' עד י"ד הם ו' והוא ממון הראשון | |
| והב' שליש וד' יהיו ו' והנה מו' עד י"ד ח' וככה ממון השני | |
| הנה בזה אמ' הראשון לשני אני אתן מה שבידי ואתה שליש מה שבידך | |
| וכן תעשה לעולם על הדרך הזה בשני אנשים | |
| ודמיון לשלשה ככה שתקח חצי העולה מן המורה כל החלקים השלשה ותדע להם המחיר והוא שתקח למשל השאלה הראשונה י"ב והוא המורה | |
| וכאשר חברנו אליו כפלו ושלישיתו מלבד שהוא חציו מלגיו והוא ו' ורביעיתו מלבד והוא שלישיתו מלגיו והם ד' וחמישיתו מלבד שהוא רביעיתו מלגיו והם ג' יעלו הכל כ"ה וחצאים י"ב וחצי וככה מחיר הדג | |
| אחר כן תוסיף חלק כל אחד מהם כפי מה שהראיתיך למעלה | |
| וכאשר רצינו לעשות כזה לד' אנשים נקח המורה שנרצה ונחבר אליו כל הד' שנרצה להוסיף עליו ומן המחובר קח השלישית והוא המחיר | |
| וכאשר תרצה לעשות זה בה' אנשים קח הרביעית ולו' קח החמישית וכן עד אין קץ | |
| שאלה שלשה אנשים רצו לקנות דג ואמרו שיתן כל אחד כמה שיש לו . אם כן כמה היה מחירו . וכמה היה לכל אחד ואחד | |
| תשובה דע כי דמי הדג דמי הדג היו י"ז פשוטים והיו לראשון ה' ולשני י"א ולשלישי י"ג | |
| וכיצד הוצאת החשבון נבקש תחלה המורה וככה תעשה תחשוב איזהו חשבון שאם תטול ממנו רביעיתו ישאר ג' מניין האנשים והנה הוא ד' | |
| אחר כן תחשוב מספר אשר תטול ממנו שלישיתו וישאר ג' תמצא שהוא ד' ומחצה | |
| אחר כך תחשוב מספר אשר תטול ממנו חציו וישאר ג' תמצא שהוא ו' | |
| וכדי שלא יהיה שברים בחשבון תכפלם ויהיה המספר הראשון ח' והשני ט' והשלישי י"ב ונמצא שעולין כ"ט | |
| עתה קח מספר האנשים שהוא ג' וחסר ממנו אחד ישארו ב' כפול הי"ב באלו הב' יעלו כ"ד תוציאם מכ"ט וישארו ה' וזהו ממון הראשון | |
| עתה כפול הט' בב' הנזכרים ויעלו י"ח תוציאם מכ"ט וישארו י"א והוא ממון השני | |
| עתה תכפול הח' בב' הנזכרים ויעלו י"ו תסירם מכ"ט ישארו י"ג והוא ממון השלישי | |
| וכולם על זה הדרך | |
| חשבון האצבעות הנקרא בערבי אל גבאר | |
| אלה שמות האצבעות גודל אצבע אמה קמיצה זרת | |
| פרק אמצעי של זרת קרוי א' | |
| אמצעי של קמיצה קרוי ב' | |
| אמצעי של אמה עמהם קרוי ג' | |
| כשתסיר הזרת קרוי ד' | |
| כשתסיר הקמיצה קרוי ה' | |
| כשתסיר האמה ותשפיל הקמיצה לבד קרוי ו' | |
| זרת שוכב קרוי ז' | |
| קמיצה עמו קרוי ח' | |
| אמה עמהם קרוי ט' | |
| הרי באלו הג' אצבעות משתמש האדם מא' ועד ט' | |
| גודל ואצבע משתמשין מי' ועד ק' | |
| כיצד יסודו של אצבע בסוף פרק אמצעי של גודל קרוי י' | |
| גודל ואצבע מחוברין זה עם זה קרוי כ' בשרשיהם זה עם זה קרוי ל' | |
| גודל על אצבע מ' | |
| גודל על הכף קרוי נ' | |
| פרק אמצעי של אצבע על פרק ראשון של גודל קרוי ס' | |
| גודל פשוט ופרק אמצעי של אצבע כנגד חודו קרוי ע' | |
| פרק אמצעי של אצבע על פרק ראשון של גודל קרוי פ' | |
| אצבע בתוך וגודל סביבו קרוי צ' | |
| עד כאן ביד ימין | |
| וביד שמאל משתמשין מאות ממאה ועד מאה אלף | |
| כיצד חודו של אצבע בסוף פרק אמצעי של גודל קרוי ק' | |
| גודל ואצבע מחוברין זה עם זה קרוי ר' | |
| שרשיהם מנשקים זה עם זה קרוי ש' | |
| גודל על אצבע קרוי ת' | |
| גודל כפוף ביד שמאל קרוי רבבה שהם י' אל אלפים | |
| כמו שחשבנו ביד ימין לאחדים כן נחשוב ביד שמאל למאות וסימניך יפול מצדך אלף ורבבה מימנך | |
| והמשכילים יזהירו כזוהר הרקיע | |
| שאלה חלק ס' על ג' בני אדם לאחד השליש ולאחד הרביע ולאחד החומש ולא ישאר מהס' | |
| תשובה נשים המורה והוא שנחבר השליש והרביע והחומש מס' הנה שליש ס' הם כ' ורביעיתם ט"ו וחמישיתם י"ב נחברם ויעלו מ"ז והוא המורה | |
| עתה כפול המספר הראשון המתחלק שהוא ס' על כל אחד מהשלשה חלקים שהם השלישית והרביעית והחמישית והעולה בכפל תחלקהו על המורה והיוצא מהחלוק הוא חלק כל אחד מהשלשה כפי החלק שיש לו לקחת | |
| וככה תעשה כפול ס' על כ' יעלו ת"ר תחלקם על מ"ז שהוא המורה ויעלו י"ג בקרוב כי הנה בדקדוק הם י"ב שלמים ול"ו שברים וזה חלק בעל השליש | |
| עתה נכפול ס' על ט"ו יעלו תת"ק נחלקם על מ"ז ויצא מן החלוק י"ט והוא חלק בעל הרביע | |
| עתה נחזור לכפול ס' על י"ב יעלו תש"כ תחלקם על מ"ז יצא בחילוק י"ח והוא חלק בעל החומש | |
| והבחינה בזה שתחבר הכל יחד ויעלו ס' | |
| וכן כל כיוצא בזה | |
| ושאלה זו יוצאה משאלת ד' אנשים שהרויחו י"ט דינרי' שהוא שאלה שנייה משער ו' | |
| שאלה ממון לקחנו ממנו שלישיתו ורביעיתו ועוד ב' ונש' ונשארו עשרה כמה הוא הממון | |
| תשובה נאמר כי השאלה היא כ"ד | |
| נקח ממנו שלישיתו ורביעיתו שהוא השליש ח' והרביע ו' עלו י"ד ונשארו י' | |
| נסיר ב' מי' ונשארו ח' ועדיין לא יצא אמתי | |
| אם כן נעשה הרביע ובתחלה נעשה המורה והוא שנכפול ג' בד' ויעלו י"ב והוא המורה | |
| נסיר מהם שלישיתו ורביעיתו שהם ז' וישארו ה' והנה יש לנו שלשה מספרים ידועים והם המורה שהוא י"ב | |
| מעתה נשים האמצעיים חברים שהם הי"ב והי"ב ונכפול זה על זה ויעלו קמ"ד נחלקם ה' ויצא בחילוק כ"ד שלמים וד' חומשים | |
| ואם תבדוק תמצאנו אמתי | |
| שאלה היו לשר אחד עשרים משרתים בין זכרים בין נקבות ונתן להם עשרים ככרי לחם ואמר להם מאלו עשרים ככרות תקחו כל א' זכר בכם ב' ככרות וכל נקבה חצי ככר כדי שיבא ככר אחד לשתי נקבות ותשאירו לי ככר לחם אחד יש לשאול כמה היו הזכרים וכמה היו הנקבות | |
| תשובה האנשים היו ששה והנשים י"ד לקחו האנשים הששה י"ב ככרות והנשי' הי"ד לקחו ז' ככרות הרי י"ט ככרות נשאר אחד לאכילת השר | |
| שאלה היו לשר אחד עשרים בהמות בין סוסים ופרדים וחמורים ונתן לעבדו עשרים עמרים של שעורים וצוה לו שיתן לכל סוס מהם ב' עמרים ולכל פרד עמר אחד ולכל חמור חצי עמר כמה בהמות היו לו מכל מין | |
| תשובה הסוסים היו ד' והפרדים היו ח' והחמורים היו ח' ונתן לד' הסוסים ב' עמרים לכל אחד הנה ח' עמרים נתן לח' פרדים עמר לפרד הנה ח' עמרים | |
|
שאלה תגר אחד אמר למשרתו הנני נותן לך ק' פשיטים קנה לי בהם ק' עופות שיהיו מג' מינים תרנוגלין ואווזים וצפרים והיה שוה האווז ה' פשיטים והתרנגול ג' פשיטים והצפרים עשרים מהם בפשוט כמה לקח מכל מין |
| תשובה מן האווזים לקח י"ח ומן התרנוגלין ב' ומן הצפרים פ' נתן בי"ח אווזים צ' פשיטים ונתן בב' תרנוגלין ו' פשיטים הנה צ"ו פשיטים וכ' עופות נתן עוד ד' פשיטים בפ' צפרים הרי ק' עופות וק' פשיטים | |
| שאלה אדם אחד אהב אשה ואמרה לו אם תתן לי תפוח אחד מגנת המלך אהיה נכבשת לך ואם לאו לא אכבש לך והלך לשוער הגן ושאל לו תפוח | |
| תשובה מאשר שאלו כל אחד מחצית שבידו וחצי תפוח יותר ושלא יתחלק שום תפוח מזה ידענו שליקט נפרדים | |
| ועתה נוציא מספרים הוא הוציא לחוץ תפוח אחד והוסיף לשוער הראשון חצי תפוח ממה שהיה בידו אם כן תפוח וחצי היה מחצית מה שנשאר לו מן השוער השני | |
| נכפלם ועולים ג' וחצי תפוח שהוסיף לשני הרי ג' וחצי וזהו החצי שנשאר לו מן השוער הפנימי | |
| נכפלם ויהיו ז' וחצי תפוח שהוסיף לשוער הפנימי הרי ז' וחצי וזהו חצי מה שליקט | |
| [נכפלם] ועולים ט"ו עתה נ | |
| כלל אם תרצה לידע תשבורת העגולה | |
| קח רביע הקו המקיף וכפול אותו על הקוטר ומה שיצא הוא תשבורת העגולה | |
| דמיון הקו המקיף שיעורו כ"ב מעלות הנה הקוטר ז' | |
| כפול ז' על ה' וחצי והנה המבוקש ל"ח וחצי והוא התשבורת | |
| וכל זה בעגולה השטחית | |
| אבל בכדור יצטרך לכפול כל הקוטר על כל הקו המקיף ואז ידע תשבורת כל הקף הכדור | |
| דמיון נכפול ז' שהוא הקוטר על כ"ב שהוא הקו המקיף ויעלה קנ"ד והוא תשבורת הכדור | |
| ולדעת שטח כל הכדור נכפול כל התשבורת בששית הקוטר ונשיג המבוקש | |
| כלל כשתרצה להוציא שורש מספר אחד ממספר אחר | |
| קח כפלו וכפל כפלו ותקח שרש העולה וחצי השורש ההוא הוא שרש מבוקשך | |
| דמיון תרצה למצוא שורש ט' | |
| קח כפלו והוא י"ח וכפל י"ח שהוא כפלו של ט' ויעלו ל"ו, תקח שרשם והוא ו' וחציו ג' וזהו שרש ט' | |
| דמיון אחר רצינו למצוא שרש י"ו | |
| קח כפלו והוא ל"ב וכפלם ועלו ס"ד ושרשם ח' וחציו ד' והוא שרש י"ו | |
| שאלה ראובן לקח משמעון בהלואה תיבה אחת מלאה חטה והיתה מכילה ח' מדות בחשבון מעוקב רוצה לומ' ח' מדות מדות באורך וח' ברוחב וח' בגובה ולקץ שנה רצה לפרוע והביא תיבה אחרת שהיתה מכילה ד' על ד' באורך וברחב ובגובה עתה נדע כמה השיב לו אם חצי החצ החטה או שלישית או רביעית | |
| ונעשה החשבון ככה נכפול ח' על ח' עלו ס"ד ונכפול ח' על ס"ד למצוא המעוקב ועלו תקי"ב והוא מה שלקח שמעון בהלואה | |
| עתה נמנה מה שהשיב לו כמה הוא נכפול ד' על ד' עלו י"ו ונכפול ד' על י"ו עלו ס"ד וזהו מה שהשיב לו | |
| עתה ניחס אותו לדעת אלו הס"ד כמה חלקים הם מתקי"ב מצאנו שהם שמיניתם ואם כן השיב לו שמינית החטה והשאר במעות | |
| ז'ל'ל ש'ל'ע' |
Notes
Apparatus
Chapter One
Chapter Two
Chapter Three
Chapter Four
Appendix I: Glossary of Terms
Appendix II: BibliographyAbraham ben Meir Ibn ‛Ezra
Manuscripts:
Edition:
Bibliography:
|


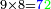
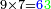
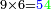


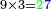
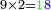
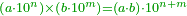
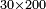
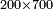
![\scriptstyle{\color{OliveGreen}{\left[\left(a\sdot10^n\right)-b\right]\times\left[\left(a\sdot10^n\right)+b\right]=\left(a\sdot10^n\right)^2-b^2}}](/mediawiki/images/math/0/e/4/0e43183966b0283d21633a6ca567a8a4.png)
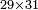
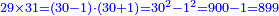
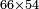
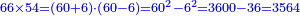

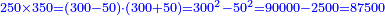
![\scriptstyle{\color{OliveGreen}{a^2=\left[10\sdot\left(\frac{1}{3}a\right)^2\right]-\left(\frac{1}{3}a\right)^2}}](/mediawiki/images/math/d/6/9/d6970429c262ac807f1717b6b1a451d1.png)

![\scriptstyle{\color{blue}{3^2=\left[10\sdot\left(\frac{1}{3}\sdot3\right)^2\right]-\left(\frac{1}{3}\sdot3\right)^2=\left(10\sdot1^2\right)-1^2=\left(10\sdot1\right)-1=10-1=9}}](/mediawiki/images/math/1/9/7/19714ab4bc2b7c48fe6734164dbdb01f.png)
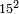
![\scriptstyle{\color{blue}{15^2=\left[10\sdot\left(\frac{1}{3}\sdot15\right)^2\right]-\left(\frac{1}{3}\sdot15\right)^2=\left(10\sdot5^2\right)-5^2=\left(10\sdot25\right)-25=250-25=225}}](/mediawiki/images/math/5/e/4/5e42240cdce4a44c236e1cb2f8813193.png)
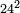
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle24^2&\scriptstyle=\left[10\sdot\left(\frac{1}{3}\sdot24\right)^2\right]-\left(\frac{1}{3}\sdot24\right)^2=\left(10\sdot8^2\right)-8^2\\&\scriptstyle=\left(10\sdot64\right)-64=640-64=576\\\end{align}}}](/mediawiki/images/math/e/6/c/e6ca108d11ec4cfb5a8e03f8aebb9c74.png)
![\scriptstyle{\color{OliveGreen}{a^2=\left[10\sdot\left[\frac{1}{3}\sdot\left(a-1\right)\right]^2\right]-\left[\frac{1}{3}\sdot\left(a-1\right)\right]^2+\left[a+\left(a-1\right)\right]}}](/mediawiki/images/math/e/6/e/e6e7d535d5d21c5c108ed87325cabeb1.png)
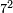
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle7^2&\scriptstyle=\left[10\sdot\left[\frac{1}{3}\sdot\left(7-1\right)\right]^2\right]-\left[\frac{1}{3}\sdot\left(7-1\right)\right]^2+\left[7+\left(7-1\right)\right]\\&\scriptstyle=\left[10\sdot\left(\frac{1}{3}\sdot6\right)^2\right]-\left(\frac{1}{3}\sdot6\right)^2+\left(7+6\right)\\&\scriptstyle=\left(10\sdot2^2\right)-2^2+13=\left(10\sdot4\right)-4+13\\&\scriptstyle=40-4+13=36+13=49\\\end{align}}}](/mediawiki/images/math/b/b/4/bb494ac822e69cc72a12d9f402a6f278.png)

![\scriptstyle{\color{blue}{\begin{align}\scriptstyle22^2&\scriptstyle=\left[10\sdot\left[\frac{1}{3}\sdot\left(22-1\right)\right]^2\right]-\left[\frac{1}{3}\sdot\left(22-1\right)\right]^2+\left[22+\left(22-1\right)\right]\\&\scriptstyle=\left[10\sdot\left(\frac{1}{3}\sdot21\right)^2\right]-\left(\frac{1}{3}\sdot21\right)^2+\left(22+21\right)\\&\scriptstyle=\left(10\sdot7^2\right)-7^2+43=\left(10\sdot49\right)-49+43\\&\scriptstyle=490-49+43=441+43=484\\\end{align}}}](/mediawiki/images/math/f/9/1/f9148c9db3895b7621089f8bdf8ab194.png)
![\scriptstyle{\color{OliveGreen}{a^2=\left[10\sdot\left[\frac{1}{3}\sdot\left(a+1\right)\right]^2\right]-\left[\frac{1}{3}\sdot\left(a+1\right)\right]^2-\left[a+\left(a+1\right)\right]}}](/mediawiki/images/math/1/d/9/1d994891701d695cd96170693d0a22b6.png)
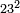
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle23^2&\scriptstyle=\left[10\sdot\left[\frac{1}{3}\sdot\left(23+1\right)\right]^2\right]-\left[\frac{1}{3}\sdot\left(23+1\right)\right]^2-\left[23+\left(23+1\right)\right]\\&\scriptstyle=\left[10\sdot\left(\frac{1}{3}\sdot24\right)^2\right]-\left(\frac{1}{3}\sdot24\right)^2-\left(23+24\right)\\&\scriptstyle=\left(10\sdot8^2\right)-8^2-47=\left(10\sdot64\right)-64-47\\&\scriptstyle=640-64-47=576-47=529\\\end{align}}}](/mediawiki/images/math/9/d/c/9dcf5ef101472d9e434643f0ca31067f.png)

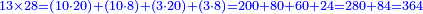
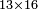
![\scriptstyle{\color{blue}{13\times16=\left[10\sdot\left(3+16\right)\right]+\left(3\sdot6\right)=\left(10\sdot19\right)+18=190+18=208}}](/mediawiki/images/math/b/6/b/b6b354e000d6d94851f660a83d6d5a43.png)
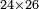
![\scriptstyle{\color{blue}{24\times26=\left[20\sdot\left(4+26\right)\right]+\left(4\sdot6\right)=\left(20\sdot30\right)+24=600+24=624}}](/mediawiki/images/math/8/f/a/8fa1c5f09d813c65541565d8773f4332.png)
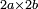
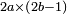
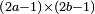
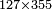
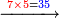
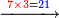
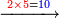
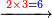
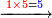






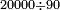


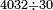




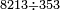

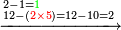
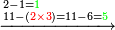

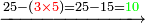
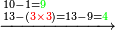



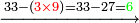
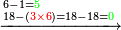

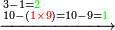
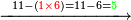


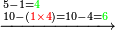
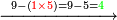

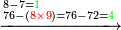
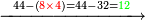
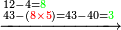

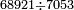

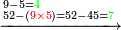
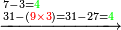
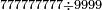
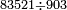
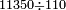
![\scriptstyle\sum_{i=1}^{n} i=n\sdot\left[\left(\frac{1}{2}\sdot n\right)+\frac{1}{2}\right]](/mediawiki/images/math/3/5/2/352391748eb2e7ace44f90cd627ef185.png)
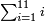
![\scriptstyle{\color{blue}{\sum_{i=1}^{11} i=11\sdot\left[\left(\frac{1}{2}\sdot11\right)+\frac{1}{2}\right]=11\sdot\left[\left(5+\frac{1}{2}\right)+\frac{1}{2}\right]=11\sdot6=6}}](/mediawiki/images/math/2/2/d/22de77a228550c8df906f2b1e8c65725.png)

![\scriptstyle{\color{blue}{\sum_{i=1}^{18} i=\left[18\sdot\left(\frac{1}{2}\sdot18\right)\right]+\left(\frac{1}{2}\sdot18\right)=\left(18\sdot9\right)+9=162+9=171}}](/mediawiki/images/math/8/7/4/874803a8411918954e47b3f65a1fc030.png)
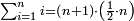
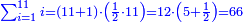
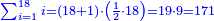
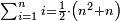
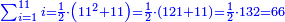
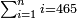
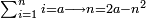

![\scriptstyle\sum_{i=1}^{n} i=\left[2\sdot\left(\frac{1}{2}\sdot n\right)^2\right]+\left(\frac{1}{2}\sdot n\right)](/mediawiki/images/math/2/6/7/2671e4a0d340332685868b16542fefd5.png)
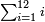
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle\sum_{i=1}^{12} i&\scriptstyle=\left[2\sdot\left(\frac{1}{2}\sdot12\right)^2\right]+\left(\frac{1}{2}\sdot12\right)\\&\scriptstyle=\left(2\sdot6^2\right)+6\\&\scriptstyle=\left(2\sdot36\right)+6=72+6=78\\\end{align}}}](/mediawiki/images/math/5/3/8/5386d11a4a2f69552f5c608d501c4696.png)
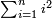
![\scriptstyle\sum_{i=1}^{n} i^2=\left(\sum_{i=1}^{n} i\right)\sdot\left[\left(\frac{2}{3}\sdot n\right)+\frac{1}{3}\right]](/mediawiki/images/math/f/3/8/f383bed5b67ee7c245135a7fb57622d9.png)
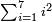
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle\sum_{i=1}^{7} i^2&\scriptstyle=\left(\sum_{i=1}^{7} i\right)\sdot\left[\left(\frac{2}{3}\sdot7\right)+\frac{1}{3}\right]\\&\scriptstyle=28\sdot\left[\left(\frac{2}{3}\sdot6\right)+\frac{2}{3}+\frac{1}{3}\right]\\&\scriptstyle=28\sdot\left(4+1\right)\\&\scriptstyle=28\sdot5=140\\\end{align}}}](/mediawiki/images/math/2/6/9/26955345db1f1607798af84338e4bc4f.png)
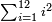
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle\sum_{i=1}^{12} i^2&\scriptstyle=\left(\sum_{i=1}^{12} i\right)\sdot\left[\left(\frac{2}{3}\sdot12\right)+\frac{1}{3}\right]\\&\scriptstyle=78\sdot\left(8+\frac{1}{3}\right)\\&\scriptstyle=\left(78\sdot8\right)+\left(\frac{1}{3}\sdot78\right)=624+26=650\\\end{align}}}](/mediawiki/images/math/5/5/7/557f6b860059c88e384ab692f9fd9cc7.png)
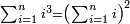

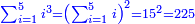
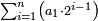

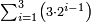
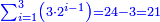

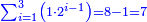
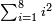
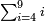
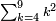
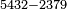
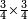
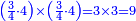
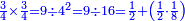

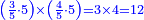


![\scriptstyle{\color{blue}{\left[\frac{2}{3}\sdot\left(3\times4\right)\right]\times\left[\frac{3}{4}\sdot\left(3\times4\right)\right]=8\times9=72=\frac{1}{2}\sdot144=\frac{1}{2}\sdot\left(3\times4\right)^2}}](/mediawiki/images/math/2/1/5/215d2c7b0742cce91ae400beb3c3806b.png)
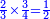
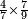
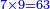
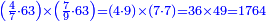


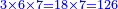
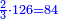

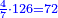
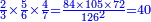
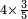
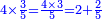
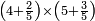
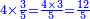
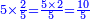

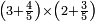

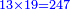

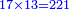
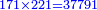
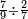
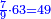

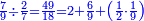
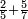
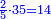
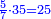

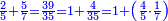

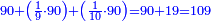

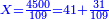

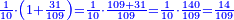

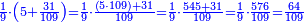
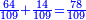
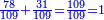
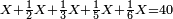
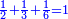
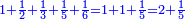
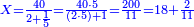
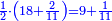
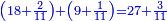
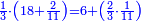
![\scriptstyle{\color{blue}{\left(27+\frac{3}{11}\right)+\left[6+\left(\frac{2}{3}\sdot\frac{1}{11}\right)\right]=33+\frac{3}{11}+\left(\frac{2}{3}\sdot\frac{1}{11}\right)}}](/mediawiki/images/math/d/2/b/d2bfd3f54a1426769648b9ae7c4c1ffb.png)


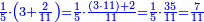

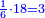
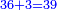

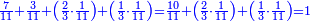
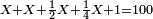
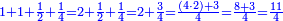
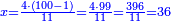
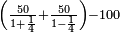
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle\left(\frac{50}{1+\frac{1}{4}}+\frac{50}{1-\frac{1}{4}}\right)-100&\scriptstyle=\left(\frac{4\sdot50}{5}+\frac{4\sdot50}{3}\right)-100\\&\scriptstyle=\left(\frac{200}{5}+\frac{200}{3}\right)-100\\&\scriptstyle=\left[40+\left(66+\frac{2}{3}\right)\right]-100\\&\scriptstyle=6+\frac{2}{3}\\\end{align}}}](/mediawiki/images/math/f/f/e/ffec10b7402d6c289bc7ae654748b4d6.png)
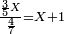
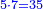
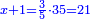
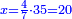
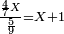


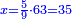
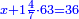

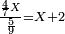

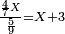

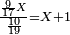

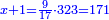
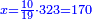
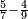
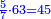
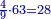

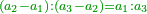

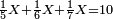

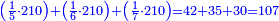
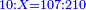
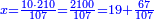
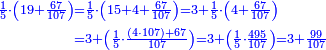
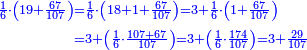
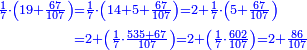
![\scriptstyle{\color{blue}{\begin{align}&\scriptstyle\left[\frac{1}{5}\sdot\left(19+\frac{67}{107}\right)\right]+\left[\frac{1}{6}\sdot\left(19+\frac{67}{107}\right)\right]+\left[\frac{1}{7}\sdot\left(19+\frac{67}{107}\right)\right]\\&\scriptstyle=\left(3+\frac{99}{107}\right)+\left(3+\frac{29}{107}\right)+\left(2+\frac{86}{107}\right)=3+3+2+2=10\\\end{align}}}](/mediawiki/images/math/b/4/8/b4826ea35cb4dcb01a63849b0817a056.png)
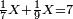
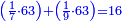
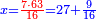
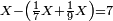
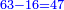
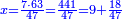
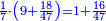
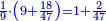
![\scriptstyle{\color{blue}{\left(9+\frac{18}{47}\right)-\left[\left(1+\frac{16}{47}\right)-\left(1+\frac{2}{47}\right)\right]=7}}](/mediawiki/images/math/2/a/5/2a59a0d2d2706fec62409829e96956f4.png)
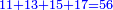



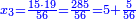
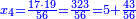
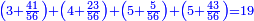
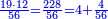
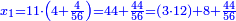
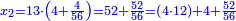
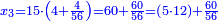
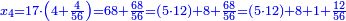
![\scriptstyle{\color{blue}{\left[\left(3\sdot12\right)+8+\frac{44}{56}\right]+\left[\left(4\sdot12\right)+4+\frac{52}{56}\right]+\left[\left(5\sdot12\right)+\frac{60}{56}\right]+\left[\left(5\sdot12\right)+8+1+\frac{12}{56}\right]=19\sdot12}}](/mediawiki/images/math/0/2/2/0229f56668a3c70d6b4891ada0ff0c48.png)
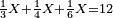
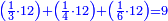
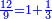
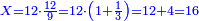
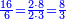
 [which are two dinar]
[which are two dinar]

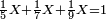
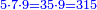
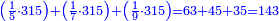
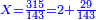
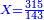
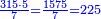
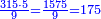

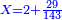
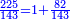
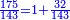
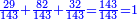
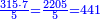
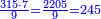
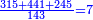
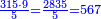
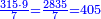

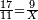
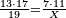
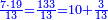

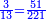
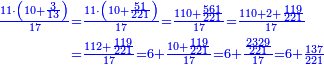

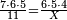


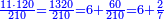
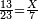
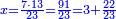
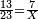
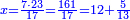
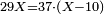
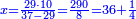
![\scriptstyle2\sdot\left[2\sdot\left[2\sdot\left(2X-2\right)-2\right]-2\right]=2](/mediawiki/images/math/a/c/0/ac095356b36a77f4fb4a1ac6de9f4dd9.png)
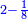
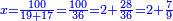
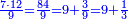
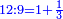
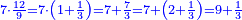
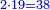

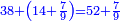
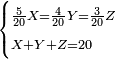
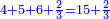
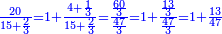
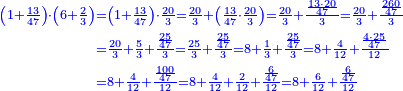
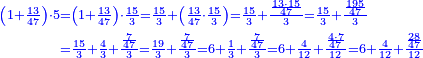
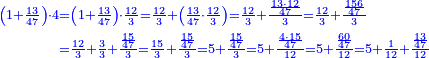
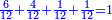


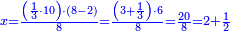
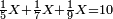

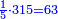
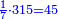
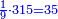
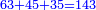
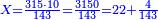
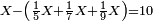
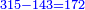
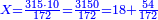
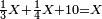
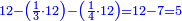
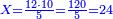
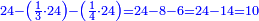

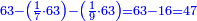
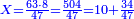
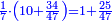
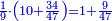
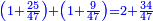
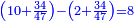
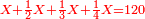
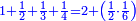
![\scriptstyle{\color{blue}{\left[2+\left(\frac{1}{2}\sdot\frac{1}{6}\right)\right]\sdot60=125}}](/mediawiki/images/math/f/e/e/feee1688775c37c50f8c73a0ca686bf5.png)
![\scriptstyle{\color{blue}{\left[2+\left(\frac{1}{2}\sdot\frac{1}{6}\right)\right]\sdot12=12+13}}](/mediawiki/images/math/c/8/4/c84d44d55e4f0aecc61dd6b809638c69.png)
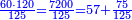
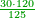
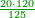


![\scriptstyle{\color{blue}{\left(\frac{1}{4}\sdot30\right)+\left[\frac{1}{3}\sdot\left(\frac{1}{4}\sdot40\right)\right]=\left(7+\frac{1}{2}\right)+\left(\frac{1}{3}\sdot10\right)=\left(7+\frac{3}{6}\right)+\left(3+\frac{2}{6}\right)=10+\frac{5}{6}}}](/mediawiki/images/math/8/0/2/8025548d7ffea180aaf74b2aa258a187.png)
![\scriptstyle{\color{blue}{\left(\frac{1}{4}\sdot30\right)+\left[\frac{1}{3}\sdot\left(\frac{1}{4}\sdot40\right)\right]+\left[\frac{1}{2}\sdot\left(\frac{1}{3}\sdot60\right)\right]=20+\frac{5}{6}}}](/mediawiki/images/math/6/3/9/639c2256db341185f9c23923c2b05f10.png)
![\scriptstyle{\color{red}{\left(\frac{1}{4}\sdot30\right)+\left[\frac{1}{3}\sdot\left(\frac{1}{4}\sdot40\right)\right]+\left[\frac{1}{2}\sdot\left(\frac{1}{3}\sdot60\right)\right]+\left(\frac{1}{2}\sdot120\right)=80+\frac{5}{6}}}](/mediawiki/images/math/2/6/5/265522ddfc53b15a08af105f276818cf.png)
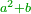 :
: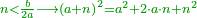
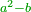 :
: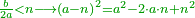

![\scriptstyle{\color{blue}{\begin{align}\scriptstyle14^2=\left(10+4\right)&\scriptstyle={\color{red}{200-\left[200-\left(10+4\right)^2\right]}}\\&\scriptstyle=200-\left[100-\left(2\sdot10\sdot4\right)-4^2\right]\\&\scriptstyle=200-\left[100-\left(20\sdot4\right)-4^2\right]\\&\scriptstyle=200-\left(100-80-16\right)\\&\scriptstyle=200-\left(20-16\right)=200-4=196\\\end{align}}}](/mediawiki/images/math/b/7/2/b7259e2f5d0b1f0f11412fa84adf0e32.png)

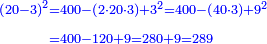
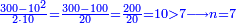
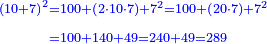
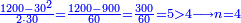
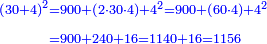

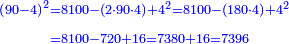
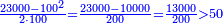
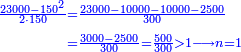
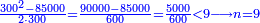



![\scriptstyle{\color{blue}{\begin{align}\scriptstyle447^2=\left(440+7\right)^2&\scriptstyle={\color{red}{200000-\left[200000-\left(440+7\right)^2\right]}}\\&\scriptstyle{\color{red}{=200000-\left[200000-440^2-\left(2\sdot440\sdot7\right)-7^2\right]}}\\&\scriptstyle=200000-\left[6400-\left(2\sdot440\sdot7\right)-7^2\right]\\&\scriptstyle=200000-\left(240-7^2\right)=200000-191=199809\\\end{align}}}](/mediawiki/images/math/6/1/c/61c58bb30da58573d3a27dd1384840c1.png)

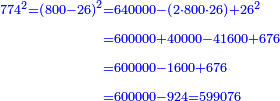
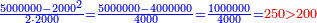
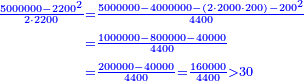

![\scriptstyle{\color{blue}{\begin{align}\scriptstyle2236^2=\left(2230+6\right)^2&\scriptstyle={\color{red}{5000000-\left[5000000-\left(2230+6\right)^2\right]}}\\&\scriptstyle{\color{red}{=5000000-\left[5000000-2230^2-\left(2\sdot2230\sdot6\right)-6^2\right]}}\\&\scriptstyle=5000000-\left[27100-\left(2\sdot2230\sdot6\right)-6^2\right]\\&\scriptstyle=5000000-\left(340-36\right)=5000000-304=4999696\\\end{align}}}](/mediawiki/images/math/2/a/6/2a64d5bea114f5b6c43277e25c9b5644.png)
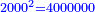




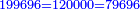


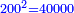





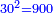

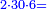
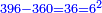
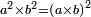
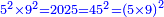
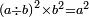

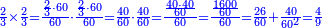
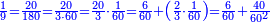
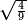
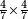
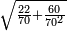
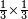
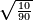
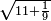
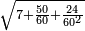
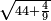
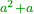
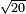
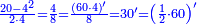
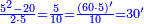
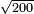

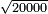

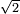
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle\sqrt{2}=\frac{1}{10}\sdot\sqrt{200}&\scriptstyle\approx\frac{1}{10}\sdot\left(14+\frac{1}{7}\right)\\&\scriptstyle=\left(\frac{1}{10}\sdot10\right)+\left(\frac{1}{10}\sdot4\right)+\left(\frac{1}{10}\sdot\frac{1}{7}\right)\\&\scriptstyle=1+\frac{4}{10}+\left[\frac{1}{10}\sdot\left(8^\prime+34^{\prime\prime}\right)\right]\\&\scriptstyle=1+\frac{2}{5}+\left(\frac{1}{10}\sdot514^{\prime\prime}\right)\approx1+24^\prime+52^{\prime\prime}\\\end{align}}}](/mediawiki/images/math/8/9/0/8901182bd27b3df60b59121aca28e18c.png)
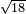
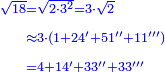
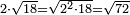


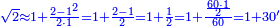

![\scriptstyle{\color{blue}{\begin{align}\scriptstyle\sqrt{2}&\scriptstyle\approx\left(1+25^\prime\right)-\frac{\left(5^\prime\right)^2}{2\sdot\left(1+25^\prime\right)}\\&\scriptstyle=\left(1+25^\prime\right)-\frac{25^{\prime\prime}}{2+50^\prime}\\&\scriptstyle=\left(1+25^\prime\right)-\frac{25^{\prime\prime}}{2+\frac{5}{6}}\\&\scriptstyle=\left(1+25^\prime\right)-\left(\frac{6\sdot25}{17}\right)^{\prime\prime}\\&\scriptstyle=\left(1+25^\prime\right)-\left(\frac{150}{17}\right)^{\prime\prime}\\&\scriptstyle=\left(1+25^\prime\right)-\left(8+\frac{14}{17}\right)^{\prime\prime}\\&\scriptstyle=\left(1+25^\prime\right)-\left[8^{\prime\prime}+\left(\frac{60\sdot14}{17}\right)^{\prime\prime\prime}\right]\\&\scriptstyle=\left(1+25^\prime\right)-\left[8^{\prime\prime}+\left(\frac{840}{17}\right)^{\prime\prime\prime}\right]\\&\scriptstyle=\left(1+25^\prime\right)-\left[8^{\prime\prime}+\left(49+\frac{7}{17}\right)^{\prime\prime\prime}\right]\\&\scriptstyle\approx\left(1+25^\prime\right)-\left(8^{\prime\prime}+49^{\prime\prime\prime}\right)=1+24^\prime+51^{\prime\prime}+11^{\prime\prime\prime}\\\end{align}}}](/mediawiki/images/math/9/1/7/9170ee4ca78c56740eea9ea949551c83.png)
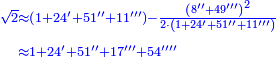

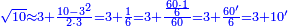
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle\sqrt{10}&\scriptstyle\approx\left(3+10^\prime\right)-\frac{\left(10^\prime\right)^2}{2\sdot\left(3+10^\prime\right)}\\&\scriptstyle=\left(3+10^\prime\right)-\frac{100^{\prime\prime}}{6+\frac{1}{3}}\\&\scriptstyle=\left(3+10^\prime\right)-\frac{3\sdot100^{\prime\prime}}{19}\\&\scriptstyle=\left(3+\frac{10}{60}\right)-\left(\frac{300}{19}\right)^{\prime\prime}\\&\scriptstyle=\left(3+10^\prime\right)-\left(15+\frac{15}{19}\right)^{\prime\prime}\\&\scriptstyle=\left(3+10^\prime\right)-\left[15^{\prime\prime}+\left(\frac{60\sdot15}{19}\right)^{\prime\prime\prime}\right]\\&\scriptstyle=\left(3+10^\prime\right)-\left[15^{\prime\prime}+\left(\frac{900}{19}\right)^{\prime\prime\prime}\right]\\&\scriptstyle=\left(3+10^\prime\right)-\left[15^{\prime\prime}+\left(47+\frac{7}{19}\right)^{\prime\prime\prime}\right]\\&\scriptstyle\approx\left(3+10^\prime\right)-\left(15^{\prime\prime}+47^{\prime\prime\prime}\right)=3+9^\prime+44^{\prime\prime}+{\color{red}{13}}^{\prime\prime\prime}\\\end{align}}}](/mediawiki/images/math/9/8/3/98331d1b1b88798e850fe18745d1155b.png)
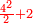
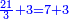
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle\sqrt{\left(\frac{1}{2}\sdot10\right)^2-\left[\left(\frac{1}{2}\sdot10\right)-1\right]^2}&\scriptstyle=\sqrt{5^2-\left(5-1\right)^2}\\&\scriptstyle=\sqrt{25-4^2}=\sqrt{25-16}=\sqrt{9}=3\\\end{align}}}](/mediawiki/images/math/8/d/6/8d69320b4b1636373c78f7298f28da0c.png)