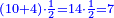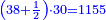Difference between revisions of "ספר החשבון והמדות"
(→Part Four: To Relate a Number To a Number) |
(→Chapter Two [on the Measuring of the Various Quadrangles]) |
||
| (423 intermediate revisions by the same user not shown) | |||
| Line 150: | Line 150: | ||
|style="text-align:right;"|והרביעי בחלוקתם | |style="text-align:right;"|והרביעי בחלוקתם | ||
|- | |- | ||
| − | |Here I start with the help of the One who grants knowledge to man. | + | |Here I start with the help of the ''One who grants knowledge to man'' [Psalms 94, 10]. |
| − | |style="text-align:right;"|ומהנה אתחיל בעזרת המלמד לאדם דעת | + | |style="text-align:right;"|ומהנה אתחיל בעזרת ''המלמד לאדם דעת''‫<ref group=note>תהילים צ"ד, י</ref> |
|- | |- | ||
|} | |} | ||
| Line 299: | Line 299: | ||
|style="text-align:right;"|וכן אל בלתי תכלית | |style="text-align:right;"|וכן אל בלתי תכלית | ||
|- | |- | ||
| − | | | + | |The total sum beneath in each of the ranks is less than ten; what is in the rank of units of the total sum are units; in the rank of the tens are tens and so on in each rank according to its position. |
|style="text-align:right;"|ואם כן יהיה הסך למטה בכל אחת מהמדרגות פחות מעשר והנמצאים בסך במדרגת האחדים הם אחדים ובמדרגת העשרות הם עשרות וכן בכל מדרגה כפי מדרגתה | |style="text-align:right;"|ואם כן יהיה הסך למטה בכל אחת מהמדרגות פחות מעשר והנמצאים בסך במדרגת האחדים הם אחדים ובמדרגת העשרות הם עשרות וכן בכל מדרגה כפי מדרגתה | ||
|- | |- | ||
| − | | | + | |If the lines you want to sum are very many, divide them into three parts, or four, or less, or more, according to the number of the lines. Do with each part by itself what was shown, then gather the total sums of all the parts and sum them up to one sum according to this same rule; the sum is the total sum of all parts together. |
|style="text-align:right;"|ואם היו הטורים אשר רצית לעשות אותם סך הרבה מאד תחלקם לשלשה חלקים או לארבעה או לפחות או ליתר לפי רוב הטורים ומיעוטם ותעשה סך לכל חלק וחלק בפני עצמו כאשר הראית אחר כן תחבר סכי כל החלקים ותעשה אותם סך כזה המשפט בעצמו ו{{#annot:term|388,1217|B2GO}}המקובץ{{#annotend:B2GO}} יהיה סך כל החלקים יחד | |style="text-align:right;"|ואם היו הטורים אשר רצית לעשות אותם סך הרבה מאד תחלקם לשלשה חלקים או לארבעה או לפחות או ליתר לפי רוב הטורים ומיעוטם ותעשה סך לכל חלק וחלק בפני עצמו כאשר הראית אחר כן תחבר סכי כל החלקים ותעשה אותם סך כזה המשפט בעצמו ו{{#annot:term|388,1217|B2GO}}המקובץ{{#annotend:B2GO}} יהיה סך כל החלקים יחד | ||
|- | |- | ||
| Line 314: | Line 314: | ||
|- | |- | ||
| | | | ||
| + | :We write them as follows: | ||
|style="text-align:right;"|כתבנו אותם סך כך | |style="text-align:right;"|כתבנו אותם סך כך | ||
|- | |- | ||
| Line 333: | Line 334: | ||
|- | |- | ||
| | | | ||
| + | :Then, we sum them up and their sum beneath is seven units, three tens, five hundreds and three thousands; so we know that they are three thousand, five hundred, and thirty-seven. | ||
|style="text-align:right;"|אחר {{#annot:term|178,1165|I96y}}חברנו אותם{{#annotend:I96y}} והיה קבוצם למטה שבע אחדים ושלש עשרות וחמש מאות ושלשת אלפים וידענו שהם שלשת אלפים וחמש מאות ושלשים ושבעה | |style="text-align:right;"|אחר {{#annot:term|178,1165|I96y}}חברנו אותם{{#annotend:I96y}} והיה קבוצם למטה שבע אחדים ושלש עשרות וחמש מאות ושלשת אלפים וידענו שהם שלשת אלפים וחמש מאות ושלשים ושבעה | ||
|- | |- | ||
| | | | ||
| + | :The numbers in the amount beneath do not reach ten in any rank. | ||
|style="text-align:right;"|ואלה המספרים בסכום היורד לא הגיעו לכלל עשר בשום מדרגה | |style="text-align:right;"|ואלה המספרים בסכום היורד לא הגיעו לכלל עשר בשום מדרגה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|ונצייר עוד צורה אחרת המגעת במדרגותיה לכלל העשרות והיא שנחבר עוד תחת אלה השני טורים שני טורים אחרים האחד תשעת אלפי' ושש מאות וחמשים ושבעה והשנית שמנת אלפים וחמש מאות וששים ושלש וזאת היא הצורה | + | *{{#annot:1215+2322+9657+8563|154|GVc4}}We draw another diagram, in which the ranks are summed up to tens, by adding two other lines beneath these two lines: one is nine thousand, six hundred, and fifty-seven; the other is eight thousand, five hundred, and sixty-three. |
| + | :<math>\scriptstyle1215+2322+9657+8563</math> | ||
| + | |style="text-align:right;"|ונצייר עוד צורה אחרת המגעת במדרגותיה לכלל העשרות והיא שנחבר עוד תחת אלה השני טורים שני טורים אחרים האחד תשעת אלפי' ושש מאות וחמשים ושבעה והשנית שמנת אלפים וחמש מאות וששים ושלש{{#annotend:GVc4}} | ||
| + | |- | ||
| + | | | ||
| + | :This is the diagram: | ||
| + | |style="text-align:right;"|וזאת היא הצורה | ||
|- | |- | ||
| | | | ||
| Line 362: | Line 371: | ||
|- | |- | ||
| | | | ||
| + | :We sum them up; the resulting sum beneath is seven units, five tens, seven hundreds, one thousand, and two tens of thousands. | ||
|style="text-align:right;"|{{#annot:term|178,1210|kqZX}}קבצנו אותם{{#annotend:kqZX}} ויצא ה{{#annot:term|388,1437|8jqg}}סך{{#annotend:8jqg}} למטה שבעה אחדים וחמש עשרות ושבע מאות ואלף אחת ושתי רבבות | |style="text-align:right;"|{{#annot:term|178,1210|kqZX}}קבצנו אותם{{#annotend:kqZX}} ויצא ה{{#annot:term|388,1437|8jqg}}סך{{#annotend:8jqg}} למטה שבעה אחדים וחמש עשרות ושבע מאות ואלף אחת ושתי רבבות | ||
|- | |- | ||
| | | | ||
| + | :When you go down their ranks on a straight line, you find the sum of the units seventeen. We subtract ten from it and write a dot in the rank of tens. Seven remains; we write it beneath the units. | ||
| + | :<math>\scriptstyle{\color{blue}{5+2+7+3=17}}</math> | ||
|style="text-align:right;"|והנה קבוץ האחדים כאשר תרד במדרגותם על קו היושר תמצאם שבעה עשר הוצאנו העשרה מהם וכתבנו נקדה אחת במדרגת העשרות ונשארו שבעה וכתבנו אותם תחת האחדים | |style="text-align:right;"|והנה קבוץ האחדים כאשר תרד במדרגותם על קו היושר תמצאם שבעה עשר הוצאנו העשרה מהם וכתבנו נקדה אחת במדרגת העשרות ונשארו שבעה וכתבנו אותם תחת האחדים | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|עוד כאשר קבצנו מדרגת העשרות על קו היורד ביושר עם הנקדה שכתבנו ‫<ref>28r</ref>שם בעד העשירית שהוצאנו מהאחדים מצאנום חמש עשרה הוצאנו עוד העשרה מהם וכתבנו נקדה בעבורם במדרגת המאות והחמשה הנשארים כתבנום למטה במדרגתן של העשרות וכן כשקבצנו המאות בירידתנו על קו היושר יחד עם הנקדה שכתבנו כנגד העשירית שהוצאנו מהעשרות מצאנום שבעה עשר הוצאנו מהם העשרה וכתבנו בעדם נקדה במדרגת האלפים והשבעה הנשארים כתבנו אותם במדרגת המאות | + | :When we sum up the rank of tens on a straight line going down, with the dot we wrote there for the ten we subtracted from the units, we find them fifteen. We subtract again ten from it and write a dot for it in the rank of hundreds. We write the remaining five beneath in the rank of tens. |
| + | :<math>\scriptstyle{\color{blue}{1+2+5+6+\sdot=15}}</math> | ||
| + | |style="text-align:right;"|עוד כאשר קבצנו מדרגת העשרות על קו היורד ביושר עם הנקדה שכתבנו ‫<ref>28r</ref>שם בעד העשירית שהוצאנו מהאחדים מצאנום חמש עשרה הוצאנו עוד העשרה מהם וכתבנו נקדה בעבורם במדרגת המאות והחמשה הנשארים כתבנום למטה במדרגתן של העשרות | ||
| + | |- | ||
| + | | | ||
| + | :When we sum up the hundreds going down on a straight line, with the dot we wrote for the ten we subtracted from the tens, we find them seventeen. We subtract ten from it and write a dot for it in the rank of thousands. We write the remaining seven in the rank of hundreds. | ||
| + | :<math>\scriptstyle{\color{blue}{2+3+6+5+\sdot=17}}</math> | ||
| + | |style="text-align:right;"|וכן כשקבצנו המאות בירידתנו על קו היושר יחד עם הנקדה שכתבנו כנגד העשירית שהוצאנו מהעשרות מצאנום שבעה עשר הוצאנו מהם העשרה וכתבנו בעדם נקדה במדרגת האלפים והשבעה הנשארים כתבנו אותם במדרגת המאות | ||
| + | |- | ||
| + | | | ||
| + | :When we sum up the thousands going down on a straight line, with the dot we wrote for the ten we subtracted from the hundreds, we find them twenty-one, which are two tens. We write two dots for them in the rank of ten thousands. We write the remaining one beneath the thousands. | ||
| + | :<math>\scriptstyle{\color{blue}{1+2+9+8+\sdot=21}}</math> | ||
| + | |style="text-align:right;"|וכן כשקבצנו את האלפים בירידתנו על קו היושר עם הנקדה שכתבנו עמם בעד העשירית שהוצאנו מהמאות מצאנו אותם עשרים ואחד הוצאנו העשרים שהם ב' עשרות וכתבנו בעבורם שתי נקדות במדרגת הרבבות והאחד הנשאר כתבנוהו תחת האלפים | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :We know from this that they are two tens of thousands, one thousand, seven hundred and fifty-seven. |
| + | |style="text-align:right;"|וידענו מזה שהם שתי רבבות ואלף אחד ושבע מאות וחמשים ושבעה | ||
|- | |- | ||
| | | | ||
| − | + | ===== <span style=color:Green>Check</span> ===== | |
| + | |||
| + | | | ||
|- | |- | ||
| | | | ||
| + | *Scales to know if your sum can be correct, by casting out nines [lit. by the Nine way]: | ||
| + | |style="text-align:right;"|<big>מאזנים</big> לדעת אם ה{{#annot:term|388,1600|Jik0}}סכום{{#annotend:Jik0}} שלך אפשר להיותו אמת על דרך התשעה | ||
| + | |- | ||
| + | | | ||
| + | :Consider all the ranks as if they are units, sum them up, then cast out the nines and keep the remainder. | ||
| + | |style="text-align:right;"|תחשוב כל המדרגו' כאלו הם אחדים ותקבצם ו{{#annot:term|1560,1265|QkW0}}תשליכם תשעה תשעה{{#annotend:QkW0}} ומה שישאר שמרהו ואם לא ישאר דבר תחשוב בלבך סיפרא | ||
| + | |- | ||
| + | | | ||
| + | :Sum up the ranks of the sum also as if they are units, then cast out the nines. If you are left with the same remainder as the former, your calculation can be correct, if the remainder is not as the former, know that you are necessarily wrong, so you should recalculate correctly. | ||
|style="text-align:right;"|עוד קבץ מדרגות הסך גם הוא כאלו הם אחדים והשליכם ט' ט' ואם {{#annot:term|936,1236|mfAW}}ישארו בידך{{#annotend:mfAW}} ההשארות כראשון אפשר שחשבונך יהיה אמת ואם לא ישארו כראשונים דע בודאי שטעית ותחזור ותחשוב בטוב | |style="text-align:right;"|עוד קבץ מדרגות הסך גם הוא כאלו הם אחדים והשליכם ט' ט' ואם {{#annot:term|936,1236|mfAW}}ישארו בידך{{#annotend:mfAW}} ההשארות כראשון אפשר שחשבונך יהיה אמת ואם לא ישארו כראשונים דע בודאי שטעית ותחזור ותחשוב בטוב | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|<big>מאזנים</big> אחרים על דרך השבעה תחשוב כל המדרגות כאשר הם ר"ל האלפים אלפים והמאות מאות והעשרות עשרות והאחדים אחדים לא שתחשבם כאלו הם אחדים כאשר עשית בדרך התשעה | + | *Other scales by casting out sevens [lit. by the Seven way]: |
| + | |style="text-align:right;"|<big>מאזנים</big> אחרים על דרך השבעה | ||
| + | |- | ||
| + | | | ||
| + | :Consider all the ranks as they are, i.e. the thousands as thousands, the hundreds as hundreds, the tens as tens, and the units as units. Do not consider them as units as you did in the way of the nines. | ||
| + | |style="text-align:right;"|תחשוב כל המדרגות כאשר הם ר"ל האלפים אלפים והמאות מאות והעשרות עשרות והאחדים אחדים לא שתחשבם כאלו הם אחדים כאשר עשית בדרך התשעה | ||
|- | |- | ||
| | | | ||
| + | :After you calculate them, cast out the sevens and keep the remainder. | ||
| + | |style="text-align:right;"|ואחר שתחשבם השליכם ז' ז' והשאר שמרהו | ||
| + | |- | ||
| + | | | ||
| + | :If nothing remains, keep a zero. | ||
| + | |style="text-align:right;"|ואם לא ישאר דבר שמור שמור בלבך סיפרא | ||
| + | |- | ||
| + | | | ||
| + | :Then, go to the ranks of the sum and start from the highest rank with the one that is next to it: consider this rank as if it is tens and the one that is next to it as units, cast out the sevens and consider the remainder as tens. Add it to the rank that is next to it and consider the further one that is next to it as units. Cast out the sevens. Do this until you arrive to the units. When you arrive to them and cast out the sevens, examine the remainder: if it is equal to what you kept, your calculation can be correct, if the it is not equal, know that you are necessarily wrong, so you should recalculate correctly. | ||
|style="text-align:right;"|אחר תבא אל מדרגות הסך ותתחיל מהכלל היותר גדול עם מה שבצדו ותחשוב כאלו הכלל הם עשרות ומה שבצדו אחדים והשליכם ז' ז' ומה שישאר תחשבהו עשרות ו{{#annot:term|178,1165|mWwI}}חברהו עם{{#annotend:mWwI}} מה שבצדו ותחשוב מה שבצדו לאחדים והשליכם ז' ז' וכן תעשה עד שתגיע אל האחדים וכשתגיע אליהם והשלכתם ז' ז' ראה הנותר ואם הוא שוה עם מה ששמרת אפשר שחשבונך יהיה אמתי ואם הוא בלתי שוה דע בודאי שטעית ותחזור ותחשוב בטוב | |style="text-align:right;"|אחר תבא אל מדרגות הסך ותתחיל מהכלל היותר גדול עם מה שבצדו ותחשוב כאלו הכלל הם עשרות ומה שבצדו אחדים והשליכם ז' ז' ומה שישאר תחשבהו עשרות ו{{#annot:term|178,1165|mWwI}}חברהו עם{{#annotend:mWwI}} מה שבצדו ותחשוב מה שבצדו לאחדים והשליכם ז' ז' וכן תעשה עד שתגיע אל האחדים וכשתגיע אליהם והשלכתם ז' ז' ראה הנותר ואם הוא שוה עם מה ששמרת אפשר שחשבונך יהיה אמתי ואם הוא בלתי שוה דע בודאי שטעית ותחזור ותחשוב בטוב | ||
|- | |- | ||
| | | | ||
| + | :Likewise, if you have divided the lines into parts, because they are many as I noted, and you have summed up part by part, do their scales like this, then sum up the scales the same way you sum up the sum in the way you know. | ||
|style="text-align:right;"|וכן אם חלקת הטורים לחלקים מצד רבויים כאשר הזכרתי ועשית הסך חלקים חלקים תעשה גם המאזנים [שלהם]‫<ref>P1031 om.</ref> ככה אחר חבר גם המאזנים ככה כמו שתחבר הסכום על הדרך אשר ידעת | |style="text-align:right;"|וכן אם חלקת הטורים לחלקים מצד רבויים כאשר הזכרתי ועשית הסך חלקים חלקים תעשה גם המאזנים [שלהם]‫<ref>P1031 om.</ref> ככה אחר חבר גם המאזנים ככה כמו שתחבר הסכום על הדרך אשר ידעת | ||
|} | |} | ||
| Line 392: | Line 443: | ||
| | | | ||
| − | ==== Chapter Three: Subtraction ==== | + | ==== Chapter Three: <span style=color:Green>Subtraction</span> ==== |
| − | |style="text-align:right;"|<big>הפרק השלישי</big> | + | |style="width:45%; text-align:right;"|<big>הפרק השלישי</big> |
|- | |- | ||
| − | | | + | |If you want to subtract a number from a number, the whole number from which you subtract must be greater than the number you subtract, this with regard to integers, but not to fractions, and for the other ranks it is not necessary [except for the highest rank]. |
|style="text-align:right;"|אם רצית לחסר מספר ממספר ראוי שיהיה כלל המספר אשר תחסר ממנו יותר גדול מהמספר אשר תחסר וזה בשלמים אך לא בשברים ובשאר המדרגות אין צורך | |style="text-align:right;"|אם רצית לחסר מספר ממספר ראוי שיהיה כלל המספר אשר תחסר ממנו יותר גדול מהמספר אשר תחסר וזה בשלמים אך לא בשברים ובשאר המדרגות אין צורך | ||
|- | |- | ||
| − | | | + | |Always write the number from which you subtract first, then write the number you subtract beneath it, each rank beneath its corresponding rank. |
|style="text-align:right;"|ולעולם תכתוב המספר אשר תחסר ממנו ראשונה אחר תכתוב תחתיו המספר אשר תחסר כל מדרגה תחת הדומה לה | |style="text-align:right;"|ולעולם תכתוב המספר אשר תחסר ממנו ראשונה אחר תכתוב תחתיו המספר אשר תחסר כל מדרגה תחת הדומה לה | ||
|- | |- | ||
| − | | | + | |If a rank is missing, write a zero in its place and always be careful not to forget the zero, but put it wherever a rank is missing. |
| − | |style="text-align:right;"|ואם יש לשם חסרון מדרגה תכתוב סיפרא במקומה ‫<ref>28v</ref>והזהר לעולם אל הסיפרא שלא תשכחנה אך בכל מקום שתחסר מדרגה תשימנה אחר כן תתחיל לחסר מהאחדים | + | |style="text-align:right;"|ואם יש לשם חסרון מדרגה תכתוב סיפרא במקומה ‫<ref>28v</ref>והזהר לעולם אל הסיפרא שלא תשכחנה אך בכל מקום שתחסר מדרגה תשימנה |
| + | |- | ||
| + | |Then, start subtracting from the units: | ||
| + | |style="text-align:right;"|אחר כן תתחיל לחסר מהאחדים | ||
|- | |- | ||
| | | | ||
| + | *If the units from which you subtract are more than those you subtract, subtract them from them and write the remainder beneath in the rank of the units. | ||
|style="text-align:right;"|ואם האחדים אשר תחסר מהם הם יותר מאלה אשר תחסר חסרם מהם והנשאר תכתוב למטה במדרגת האחדים | |style="text-align:right;"|ואם האחדים אשר תחסר מהם הם יותר מאלה אשר תחסר חסרם מהם והנשאר תכתוב למטה במדרגת האחדים | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|ואם הם | + | *If they are less than them, go to the tens next to them, take one ten, subtract it from the tens and add it to the units, then subtract from them what you subtract and write the remainder beneath in the rank of the units. |
| + | |style="text-align:right;"|ואם הם פחות מהם תחזור אל העשרות אשר בצדם וקח עשירית אחת וחסרה מן העשרות וחברה עם האחדים אחר גרע מהם מה שתגרע והשאר תכתבם למטה במדרגת האחדים | ||
| + | |- | ||
| + | |Then subtract the tens from the tens: | ||
| + | |style="text-align:right;"|עוד תבוא לחסר העשרות מהעשרות | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *If the tens from which you subtract are more [than those you subtract], subtract from them and write the remainder beneath the tens. |
| + | |style="text-align:right;"|ואם יותר העשרות שתחסר מהם חסר מהם והשאר כתבם תחת העשרות | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|ואם הם מעטים שוב אל המאות ועשה כמשפט עד שתגיע אל הכלל היותר גדול | + | *If they are less, go to the hundreds. |
| + | |style="text-align:right;"|ואם הם מעטים שוב אל המאות | ||
| + | |- | ||
| + | |Proceed according to the rule, until you reach the highest rank. | ||
| + | |style="text-align:right;"|ועשה כמשפט עד שתגיע אל הכלל היותר גדול | ||
|- | |- | ||
| − | | | + | |If there is a zero there and you want to subtract from it what is beneath it, take [one] from the rank that is higher than it. |
| − | |style="text-align:right;"|אמנם אם יש לשם סיפרא ותרצה לחסר ממנה מה שלמטה ממנה | + | |style="text-align:right;"|אמנם אם יש לשם סיפרא ותרצה לחסר ממנה מה שלמטה ממנה תקח מהכלל אשר למעלה ממנה |
|- | |- | ||
| − | | | + | |This is the diagram: |
|style="text-align:right;"|וזאת היא הצורה | |style="text-align:right;"|וזאת היא הצורה | ||
|- | |- | ||
| Line 445: | Line 509: | ||
|- | |- | ||
| | | | ||
| + | :We subtract five from four: since four is smaller than it, we go to the tens that are higher and we find one ten. We take it and make it a ten. We add it to the four; thay become fourteen. We subtract five from it; nine remains. We write it in the rank of the units. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(4+10\right)-5=14-5=9}}</math> | ||
|style="text-align:right;"|הוצאנו החמשה מן הארבעה ומפני שהארבעה פחותים מהם חזרנו אל העשרות אשר למעלה מהם ומצאנו עשירית אחת ולקחנוה ועשינוה עשרה וחברנוה עם הארבעה ונהיו ארבעה עשר הוצאנו מהם החמשה ו{{#annot:term|936,1236|bl2G}}נשארו{{#annotend:bl2G}} תשעה וכתבנום במדרגת האחדים | |style="text-align:right;"|הוצאנו החמשה מן הארבעה ומפני שהארבעה פחותים מהם חזרנו אל העשרות אשר למעלה מהם ומצאנו עשירית אחת ולקחנוה ועשינוה עשרה וחברנוה עם הארבעה ונהיו ארבעה עשר הוצאנו מהם החמשה ו{{#annot:term|936,1236|bl2G}}נשארו{{#annotend:bl2G}} תשעה וכתבנום במדרגת האחדים | ||
|- | |- | ||
| | | | ||
| + | :We want to subtract twenty from the zero, because nothing remains from the tens. We go to the hundreds, there is one hundred. We take it and make it ten tens. We subtract twenty from them; eighty remain. We write them beneath. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(0+100\right)-20=100-20=80}}</math> | ||
|style="text-align:right;"|עוד רצינו לגרוע העשרים מן הסיפרא כי לא נשאר דבר מהעשרות וחזרנו אל המאות והיה מאה אחת ולקחנוה והחזרנוה אל עשר עשרות והוצאנו ממנה העשרים ונשארו שמנים וכתבנום למטה | |style="text-align:right;"|עוד רצינו לגרוע העשרים מן הסיפרא כי לא נשאר דבר מהעשרות וחזרנו אל המאות והיה מאה אחת ולקחנוה והחזרנוה אל עשר עשרות והוצאנו ממנה העשרים ונשארו שמנים וכתבנום למטה | ||
|- | |- | ||
| | | | ||
| + | :We want to subtract two hundred from the zero, because from the hundreds we have nothing left. We go to the thousands and take one thousand from the thousands; four thousand remain. We make ten hundrad of the thousand we took. We subtract two hundred from them; eight hundred remain. We write them in the rank of the hundreds. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(0+1000\right)-200=1000-200=800}}</math> | ||
|style="text-align:right;"|עוד רצינו לגרוע המאתים מהסיפרא כי לא נשאר לנו במאות דבר וחזרנו אל האלפים ולקחנו אלף אחת מן האלפים ונשארו האלפים ד' והאלף אשר לקחנו החזרנוה עשר מאות וגרענו מהם המאתים ונשארו שמנה מאות וכתבנום במדרגת המאות | |style="text-align:right;"|עוד רצינו לגרוע המאתים מהסיפרא כי לא נשאר לנו במאות דבר וחזרנו אל האלפים ולקחנו אלף אחת מן האלפים ונשארו האלפים ד' והאלף אשר לקחנו החזרנוה עשר מאות וגרענו מהם המאתים ונשארו שמנה מאות וכתבנום במדרגת המאות | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|עוד גרענו הד' אלפים מד' אלפים ונשאר 0' | + | :We also subtract four thousand from four thousand; 0 remains. |
| + | :<math>\scriptstyle{\color{blue}{4000-4000=0}}</math> | ||
| + | |style="text-align:right;"|עוד גרענו הד' אלפים מד' אלפים ונשאר 0‫' | ||
|- | |- | ||
| | | | ||
| + | :Therefore, we know that when we subtract 4225 from 5114, zero thousands remain, and eight hundred, and eighty-nine. | ||
|style="text-align:right;"|אם כן ידענו שכשחסרנו ד' אלפים ורכ"ה מה' אלפים וקי"ד נשארו סך סיפרא אלפים ושמנה מאות ושמנים ותשעה | |style="text-align:right;"|אם כן ידענו שכשחסרנו ד' אלפים ורכ"ה מה' אלפים וקי"ד נשארו סך סיפרא אלפים ושמנה מאות ושמנים ותשעה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|<big>מאזנים</big> על דרך התשעה תחשוב כל | + | |
| + | ===== <span style=color:Green>Check</span> ===== | ||
| + | |||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | *Scales by casting out nines [lit. by the Nine way]: | ||
| + | |style="text-align:right;"|<big>מאזנים</big> על דרך התשעה | ||
| + | |- | ||
| + | | | ||
| + | :Consider all the ranks, from which you have subtracted, as if they are units, cast out the nines and keep what remains; it is called the first reserved. | ||
| + | |style="text-align:right;"|תחשוב כל המדרגות שגרעת מהם כאלו הם אחדים ותשליכם ט' ט' ומה שישאר שמרהו ויקרא השמור הראשון | ||
|- | |- | ||
| | | | ||
| + | :Do the same with the number you have subtracted; keep the remainder from the subtraction of the nines; it is called the second reserved. | ||
|style="text-align:right;"|וכן תעשה במספרים אשר גרעת אותם שתשמור מהם הנשאר מהט' ט' וזה יקרא השמור השני | |style="text-align:right;"|וכן תעשה במספרים אשר גרעת אותם שתשמור מהם הנשאר מהט' ט' וזה יקרא השמור השני | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|אחר תגרע השמור השני | + | :Then, subtract the second reserved from the first and keep what remains; it is called the third reserved. |
| + | |style="text-align:right;"|אחר תגרע השמור השני מהראשון ומה שישאר שמרהו וזה יקרא השמור השלישי | ||
| + | |- | ||
| + | | | ||
| + | :Examine also the number that remained after you have subtracted the numbers from the numbers: consider [the ranks] as if they are units and cast out the nines. If the remainder is equal to the third reserved, your calculation can be correct. If it is not equal, know that you were wrong for sure. | ||
| + | |style="text-align:right;"|עוד תעיין אל הסך הנשאר אחר שגרעת המספרים מהמספרים ותחשבם כאלו הם אחדים והשליכם ט' ט' והנשאר אם יהיה שוה לשמור השלישי אפשר שחשבונך יהיה אמת ואם אינו שוה דע בודאי שטעית | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :If the first reserved is less than the second reserved, add 9 to it, then subtract the second reserved from it and proceed as the rule. |
| + | |style="text-align:right;"|ואם השמור הראשון פחות מהשמור [השני]‫<ref>P1031: הראשון</ref> תוסיף עליו ט' אחר תגרע השמור השני ממנו ותעשה כמשפט | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *Other scales by casting out sevens [lit. by the Seven way]: |
| + | |style="text-align:right;"|<big>מאזנים</big> אחרים על דרך השבעה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Consider the ranks of the number, from which you have subtracted, as tens and those that are next to them as units. Cast out the sevens. Consider what remains as tens and the number that precedes it as units. Cast out the sevens, [and so on] until you reach the units. Cast out the sevens and keep what remains; it is called the first reserved. |
| + | |style="text-align:right;"|תחשוב כללי המספרים אשר גרעת מהם עשרות ‫<ref>29r</ref>ואשר בצדם אחדים והשליכם ז' ז' ומה שישאר תחשבהו עשרות והמספר הבא אחריו אחדים והשליכם ז' ז' עד שתגיע אל האחדים והשליכם ז' ז' ומה שישאר שמרהו וזה יקרא השמור הראשון | ||
|- | |- | ||
| | | | ||
| + | :Do the same with the number you have subtracted and keep the remainder; it is called the second reserved. | ||
|style="text-align:right;"|עוד תעשה ככה במספרים אשר גרעת אותם והנשאר שמרהו ויקרא השמור השני | |style="text-align:right;"|עוד תעשה ככה במספרים אשר גרעת אותם והנשאר שמרהו ויקרא השמור השני | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|גרע השמור השני מהשמור הראשון ואשר ישאר יקרא השמור השלישי | + | :Subtract the second reserved from the first reserved and what remains is called the third reserved. |
| + | |style="text-align:right;"|גרע השמור השני מהשמור הראשון ואשר [ישאר]‫<ref>P1031 om.</ref> יקרא השמור השלישי | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Then, examine the number that remained after you have subtracted the numbers from the numbers: consider [the ranks] as we have said concerning the first lines, meaning consider the ranks as tens and those that are next to them as units and cast out the sevens. Consider the remainder as tens and what is next to it as units and cast out the sevens. [And so on] until you reach the units. After you cast the sevens from it, examine the remainder: if it is equal to the third reserved, your calculation can be correct, otherwise, know that you were wrong for sure. |
| + | |style="text-align:right;"|אח"כ תעיין אל המספרים אשר נשארו כשגרעת המספרים מהמספרים וחשבם ככה כאשר אמרנו בטורים הראשונים כלומר כשתחשוב הכללים עשרות ואשר בצדם אחדים והשליכם ז' ז' והנשאר תחשבהו עשרות ואשר בצדו לאחדים ותשליכם ז' ז' עד שתגיע אל האחדים ואחר שהשלכתם ז' ז' עיין בנשארים ואם הם שוים לשמור השלישי אפשר שחשבונך יהיה אמת ואם לא דע כי בודאי טעית | ||
|- | |- | ||
| | | | ||
| + | *Other scales by way of addition and they are scales of truth: | ||
|style="text-align:right;"|<big>מאזנים</big> אחרים על דרך הקבוץ והם מאזני צדק | |style="text-align:right;"|<big>מאזנים</big> אחרים על דרך הקבוץ והם מאזני צדק | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|חבר המספרים שגרעת אותם עם המספרים שנשארו אחר הגרעון וראה ואם יהיו שוים עם המספרים אשר גרעת מהם תדע בודאי כי חשבונך אמת ואם | + | :Add the number you have subtracted to the number that remained after the subtraction, if they are equal to the number, from which you have subtracted, know that your calculation is correct for sure, if not, you were wrong for sure. |
| + | |style="text-align:right;"|חבר המספרים שגרעת אותם עם המספרים שנשארו אחר הגרעון וראה ואם יהיו שוים עם המספרים אשר גרעת מהם תדע בודאי כי חשבונך אמת ואם לא בודאי טעית | ||
|- | |- | ||
| − | | | + | |The first part is complete. |
| − | |style="text-align:right;"|תם החלק הראשון | + | |style="text-align:right;"|‫[תם החלק הראשון]‫<ref>P1031 om.</ref> |
|- | |- | ||
|} | |} | ||
| Line 517: | Line 615: | ||
|- | |- | ||
|Then, he takes for each the value of its rank: if the one is of the tens and the other is of the hundreds, we take two for the tens and three for the hundreds. We sum them; it is five. | |Then, he takes for each the value of its rank: if the one is of the tens and the other is of the hundreds, we take two for the tens and three for the hundreds. We sum them; it is five. | ||
| − | |style="text-align:right;"|ואח"כ יקח לכל האחד מספר במדרגתו שאם היה | + | |style="text-align:right;"|ואח"כ יקח לכל האחד מספר במדרגתו שאם היה הא' עשרות והאחר מאות נקח בעד העשרות שנים ובעד המאות שלשה ונחברם ויהיו חמשה |
|- | |- | ||
|We always subtract one and the remainder indicates for us of what rank [the product] is. | |We always subtract one and the remainder indicates for us of what rank [the product] is. | ||
| Line 525: | Line 623: | ||
*{{#annot:80×500|156|x20n}}Example: we wish to multiply eighty by five hundred. | *{{#annot:80×500|156|x20n}}Example: we wish to multiply eighty by five hundred. | ||
:<math>\scriptstyle80\times500</math> | :<math>\scriptstyle80\times500</math> | ||
| − | |style="text-align:right;"|<big>דמיון</big> | + | |style="text-align:right;"|<big>דמיון</big> בזה רצינו לכפול שמנים על חמש מאות{{#annotend:x20n}} |
|- | |- | ||
| | | | ||
| Line 550: | Line 648: | ||
|- | |- | ||
|We start multiplying the units of the bottom [line] by the units of the upper [line]. | |We start multiplying the units of the bottom [line] by the units of the upper [line]. | ||
| − | |style="text-align:right;"|ונתחיל לכפול אחדי התחתון על אחדי העליון | + | |style="text-align:right;"|ונתחיל לכפול אחדי התחתון על אחדי ‫<ref>29v</ref>העליון |
|- | |- | ||
|We write the result beneath in the rank of units, if it does not reach tens. | |We write the result beneath in the rank of units, if it does not reach tens. | ||
| Line 559: | Line 657: | ||
|- | |- | ||
|We also multiply the units below by the rank of tens above. | |We also multiply the units below by the rank of tens above. | ||
| − | |style="text-align:right;"|עוד נכפול האחדים שלמטה עם מדרגת | + | |style="text-align:right;"|עוד נכפול האחדים שלמטה עם מדרגת העשרו' שלמעלה |
|- | |- | ||
|We add the number of tens we kept to the units of this product, because although they are tens, the result is as if they are tens and units, for we multiply everything as units. | |We add the number of tens we kept to the units of this product, because although they are tens, the result is as if they are tens and units, for we multiply everything as units. | ||
| − | |style="text-align:right;"|ואשר יהיה נחבר גם מספר העשרות ששמרנו עם אחדי זה הכפל כי אע"פ שהם | + | |style="text-align:right;"|ואשר יהיה נחבר גם מספר העשרות ששמרנו עם אחדי זה הכפל כי אע"פ שהם עשרו' אבל מה שיצא מהם יהיה כאלו הם עשרות ואחדים כי על דמיון האחדים אנו כופלים הכל |
|- | |- | ||
|We write the units resulting from this multiplication in the rank of tens and we write the tens in the third rank. | |We write the units resulting from this multiplication in the rank of tens and we write the tens in the third rank. | ||
| Line 614: | Line 712: | ||
:*We multiply 5 by 3; it is 15. | :*We multiply 5 by 3; it is 15. | ||
::<math>\scriptstyle{\color{blue}{5\sdot3=15}}</math> | ::<math>\scriptstyle{\color{blue}{5\sdot3=15}}</math> | ||
| − | |style="text-align:right;"|כפלנו | + | |style="text-align:right;"|כפלנו על הג' הה' ונהיו ט"ו |
|- | |- | ||
| | | | ||
::We subtract the ten and keep it; 5 remains. We write it in the rank of units. | ::We subtract the ten and keep it; 5 remains. We write it in the rank of units. | ||
| − | |style="text-align:right;"|גרענו העשירית ושמרנוה נשארו ה' | + | |style="text-align:right;"|גרענו העשירית ושמרנוה נשארו ה' וכתבנו אותם במדרגת האחדים |
|- | |- | ||
| | | | ||
| Line 626: | Line 724: | ||
|- | |- | ||
| | | | ||
| − | ::Since no units were received, we write the ten we kept | + | ::Since no units were received, we write the ten we kept in the place of the tens and we write the twenty in the third rank. |
| − | |style="text-align:right;"|ומפני שלא יצאו מזה אחדים כתבנו העשירית האחת | + | |style="text-align:right;"|ומפני שלא יצאו מזה אחדים כתבנו העשירית האחת ששמרנו במקום העשרות והעשרים כתבנום במדרגה השלישית |
|- | |- | ||
| | | | ||
| Line 636: | Line 734: | ||
| | | | ||
::Since no units were received here also, we write zero in the place of the units and we write the six in the place of the tens. | ::Since no units were received here also, we write zero in the place of the units and we write the six in the place of the tens. | ||
| − | |style="text-align:right;"|ומפני שלא יצאו גם בכאן אחדים כתבנו במקום האחדים סיפרא והששה כתבנום במקום העשרות | + | |style="text-align:right;"|ומפני שלא יצאו גם בכאן אחדים כתבנו במקום [האחדים]‫<ref>P1031 העשרים</ref> סיפרא והששה כתבנום במקום העשרות |
|- | |- | ||
| | | | ||
| Line 645: | Line 743: | ||
| | | | ||
::We write it in the third rank, because each of the multiplicands is in the second rank and when subtracting one, three remains. | ::We write it in the third rank, because each of the multiplicands is in the second rank and when subtracting one, three remains. | ||
| − | |style="text-align:right;"|וכתבנום במדרגה השלישית כי כל אחד מהכפולים היו | + | |style="text-align:right;"|וכתבנום במדרגה השלישית כי כל אחד מהכפולים היו ממדרגת שנית ובהשלכת האחד נשארו שלשה |
|- | |- | ||
| | | | ||
| Line 711: | Line 809: | ||
:*We multiply 3 by 8; it is 24. | :*We multiply 3 by 8; it is 24. | ||
::<math>\scriptstyle{\color{blue}{3\sdot8=24}}</math> | ::<math>\scriptstyle{\color{blue}{3\sdot8=24}}</math> | ||
| − | |style="text-align:right;"|עוד כפלנו הג' | + | |style="text-align:right;"|עוד כפלנו הג' עם הח' ונהיו כ"ד |
|- | |- | ||
| | | | ||
| Line 724: | Line 822: | ||
| | | | ||
::We keep the ten and sum the two tens we kept with its units that are six; it is 8. We write it in the rank of hundreds and we write the ten we kept in the rank of thousands. | ::We keep the ten and sum the two tens we kept with its units that are six; it is 8. We write it in the rank of hundreds and we write the ten we kept in the rank of thousands. | ||
| − | |style="text-align:right;"|שמרנו העשירית האחת וחברנו עם אחדיו שהם הששה את | + | |style="text-align:right;"|שמרנו העשירית האחת וחברנו עם אחדיו שהם הששה את שתי עשרות‫<ref>P1031 אעשרות</ref> שהיו שמורות לנו ונהיו ח' וכתבנום במדרגת המאות והעשירית האחת אשר שמרנו עתה כתבנוה במדרגת האלפים |
|- | |- | ||
| | | | ||
| Line 737: | Line 835: | ||
:*We multiply it first by 5; it is twenty. | :*We multiply it first by 5; it is twenty. | ||
::<math>\scriptstyle{\color{blue}{4\sdot5=20}}</math> | ::<math>\scriptstyle{\color{blue}{4\sdot5=20}}</math> | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ונכפול תחלה עם הה' ונהיו עשרים |
|- | |- | ||
| | | | ||
| Line 750: | Line 848: | ||
| | | | ||
::We keep the ten and sum the two tens we kept with the two that are its units; it is 4. We write it in the rank of hundreds, because the numbers we multiply are of the second rank. | ::We keep the ten and sum the two tens we kept with the two that are its units; it is 4. We write it in the rank of hundreds, because the numbers we multiply are of the second rank. | ||
| − | |style="text-align:right;"|שמרנו העשירית האחת וחברנו עם השנים שהם אחדיו השני עשרות שהיו שמורות לנו ונהיו ד' כתבנום במדרגת המאות לפי שהמספרים שכפלנו הם במדרגה השניה | + | |style="text-align:right;"|שמרנו העשירית האחת וחברנו ‫<ref>30r</ref>עם השנים שהם אחדיו השני עשרות שהיו שמורות לנו ונהיו ד' כתבנום במדרגת המאות לפי שהמספרים שכפלנו הם במדרגה השניה |
|- | |- | ||
| | | | ||
| Line 759: | Line 857: | ||
| | | | ||
::We add also the ten we kept to it; it is 9. We write it in the rank of thousands, because the numbers we multiply are of the second and third ranks. | ::We add also the ten we kept to it; it is 9. We write it in the rank of thousands, because the numbers we multiply are of the second and third ranks. | ||
| − | |style="text-align:right;"|חברנו גם העשירית השמורה לנו עמם ונהיו ט' כתבנום במדרגת האלפים לפי | + | |style="text-align:right;"|חברנו גם העשירית השמורה לנו עמם ונהיו ט' כתבנום במדרגת האלפים לפי שהמספרי' אשר כפלנו היו ממדרגה השנית והשלישית |
|- | |- | ||
| | | | ||
| Line 767: | Line 865: | ||
| | | | ||
:We multiply 3 by each of the three upper digits: | :We multiply 3 by each of the three upper digits: | ||
| − | |style="text-align:right;"|עוד נשוב לכפול הג' עם כל אחד ואחד מן שלשת המספרים העליונים | + | |style="text-align:right;"|עוד נשוב לכפול הג' עם כל אחד ואחד [מן]‫<ref>P1031 הן</ref> שלשת המספרים העליונים |
|- | |- | ||
| | | | ||
| Line 794: | Line 892: | ||
| | | | ||
::We add also the reserved ten to it; it is 7. We write it in the rank of tens of thousands, because these two numbers are in the third rank. | ::We add also the reserved ten to it; it is 7. We write it in the rank of tens of thousands, because these two numbers are in the third rank. | ||
| − | |style="text-align:right;"|חברנו גם העשירית השמורה עמם ונהיו ז' וכתבנום במדרגת הרבבות כי שני המספרים האלה | + | |style="text-align:right;"|חברנו גם העשירית השמורה עמם ונהיו ז' וכתבנום במדרגת הרבבות כי שני המספרים האלה במדרגה השלישית |
|- | |- | ||
| | | | ||
:Then, we sum up the three amounts according to the rule; it is 81 thousand, seven hundred and eighty. | :Then, we sum up the three amounts according to the rule; it is 81 thousand, seven hundred and eighty. | ||
| − | |style="text-align:right;"|אחרי כן קבצנו שלשה | + | |style="text-align:right;"|אחרי כן קבצנו שלשה הסכים כמשפט ונהיו פ"א אלף ושבע מאות ושמנים |
|- | |- | ||
| | | | ||
| Line 871: | Line 969: | ||
| | | | ||
::Since there are no units here, we write the two reserved tens in the rank of tens and keep this ten. | ::Since there are no units here, we write the two reserved tens in the rank of tens and keep this ten. | ||
| − | |style="text-align:right;"|ומפני שאין שם אחדים כתבנו הב' עשרות השמורות במדרגת העשרות וזאת העשירית שמרנוה | + | |style="text-align:right;"|ומפני שאין שם אחדים כתבנו [הב' עשרות השמורות במדרגת העשרות וזאת העשירית שמרנוה |
|- | |- | ||
| | | | ||
| Line 880: | Line 978: | ||
| | | | ||
::Since there are no units here, we write the ten we kept beneath in the rank of hundreds and keep this ten in the rank of thousands. | ::Since there are no units here, we write the ten we kept beneath in the rank of hundreds and keep this ten in the rank of thousands. | ||
| − | |style="text-align:right;"|ומפני שאין שם אחדים כתבנו למטה העשירית אשר שמרנו במדרגת המאות וזאת העשירית במדרגת האלפים | + | |style="text-align:right;"|ומפני שאין שם אחדים כתבנו]‫<ref>P1031 om.</ref> למטה העשירית אשר שמרנו במדרגת המאות וזאת העשירית במדרגת האלפים |
|- | |- | ||
| | | | ||
:We multiply again each of the upper digits by zero and write zero in the rank of tens, in the rank of hundreds, and in the rank of thousands. | :We multiply again each of the upper digits by zero and write zero in the rank of tens, in the rank of hundreds, and in the rank of thousands. | ||
| − | |style="text-align:right;"|עוד שבנו להכות כל | + | |style="text-align:right;"|עוד שבנו להכות כל אחת משלשה המספרים העליונים עם הסיפרא וכתבנו סיפרא במדרגת העשרות וכן במדרגת המאות וכן במדרגת האלפים |
|- | |- | ||
| | | | ||
| Line 893: | Line 991: | ||
| | | | ||
::We write it in the rank of hundreds, because the two multiplied numbers are in the first and third ranks. | ::We write it in the rank of hundreds, because the two multiplied numbers are in the first and third ranks. | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כתבנו אותו במדרגת המאות כי שני המספרים הנכפלים היו מהמדרגה הראשונה והשלישי‫' |
|- | |- | ||
| | | | ||
| Line 902: | Line 1,000: | ||
| | | | ||
::We write it in the rank of thousands, because one of the two multiplied numbers is in the second rank and the other in the third rank. | ::We write it in the rank of thousands, because one of the two multiplied numbers is in the second rank and the other in the third rank. | ||
| − | |style="text-align:right;"|וכתבנום במדרגת האלפים כי שני | + | |style="text-align:right;"|וכתבנום במדרגת האלפים כי שני המספרי' הכפולי' היה האחד מהמדרגה השנית והאחר מהמדרגה השלישית |
|- | |- | ||
| | | | ||
| Line 911: | Line 1,009: | ||
| | | | ||
::We write it in the rank of tens of thousands, because each of two multiplied numbers is in the third rank. | ::We write it in the rank of tens of thousands, because each of two multiplied numbers is in the third rank. | ||
| − | |style="text-align:right;"|וכתבנום במדרגת הרבבות כי שני המספרים הכפולים היה כל | + | |style="text-align:right;"|וכתבנום במדרגת הרבבות כי שני המספרים הכפולים היה כל א' מהמדרגה השלישית |
|- | |- | ||
| | | | ||
| Line 917: | Line 1,015: | ||
|style="text-align:right;"|אחר כן קבצנו העולה מהם והם כ"ג אלף וחמש מאות ועשרים | |style="text-align:right;"|אחר כן קבצנו העולה מהם והם כ"ג אלף וחמש מאות ועשרים | ||
|- | |- | ||
| − | | | + | |Said Mordecai: I saw fit to teach you another easier way I have found among the Arab sages, in which you write the whole product in one line without the need for more than that. |
| − | |style="text-align:right;"|<big>אמר מרדכי</big> ראיתי להודיעך הכפל הזה בדרך אחרת נקלה מצאתיה אצל חכמי | + | |style="text-align:right;"|<big>אמר מרדכי</big> ראיתי להודיעך הכפל הזה בדרך אחרת נקלה מצאתיה אצל חכמי הישמעאלים והוא שתעשה כל סך הנכפלים בשטה אחת מבלתי שתצטרך אל יותר מזה |
| + | |- | ||
| + | |Do as follows: | ||
| + | |style="text-align:right;"|וככה תעשה | ||
|- | |- | ||
| − | | | + | |Write the number you want to multiply in the upper line as usual. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|תשים המספר אשר תרצה לכפול עליו בשטה העליונה כמנהג |
|- | |- | ||
| − | | | + | |Write also the number by which you want to multiply it in the bottom line, each rank corresponding to its similar [rank]. |
|style="text-align:right;"|עוד שים המספר אשר תרצה לכפול אותו עליו בשטה השפלה כל מדרגה כנגד הדומה לה | |style="text-align:right;"|עוד שים המספר אשר תרצה לכפול אותו עליו בשטה השפלה כל מדרגה כנגד הדומה לה | ||
|- | |- | ||
|Then, start to multiply the units by the units and write the result below. | |Then, start to multiply the units by the units and write the result below. | ||
| − | |style="text-align:right;"|ואחר כן החל לכפול האחדים על האחדים ותכתוב העולה למטה | + | |style="text-align:right;"|ואחר כן החל לכפול האחדים על האחדים ותכתוב ‫<ref>30v</ref>העולה למטה |
|- | |- | ||
|If tens are received, keep them and write only the units below. | |If tens are received, keep them and write only the units below. | ||
| − | |style="text-align:right;"|ואם יעלו עשרות תפוש אותם בלבך ותכתוב האחדים לבדם למטה | + | |style="text-align:right;"|‫[ואם יעלו עשרות תפוש אותם בלבך ותכתוב האחדים לבדם למטה]‫<ref>P1031 om.</ref> |
|- | |- | ||
|Multiply also the units that are in the bottom line by the tens that are in the upper line as if they are units by units. | |Multiply also the units that are in the bottom line by the tens that are in the upper line as if they are units by units. | ||
|style="text-align:right;"|עוד תכפול האחדים שבשטה השפלה עם העשרות שבשטה העליונה כאלו הם אחדים על אחדים | |style="text-align:right;"|עוד תכפול האחדים שבשטה השפלה עם העשרות שבשטה העליונה כאלו הם אחדים על אחדים | ||
|- | |- | ||
| − | | | + | |If you are left with tens from the first product, consider them as units and add them to these. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואם נשארו לך עשרות מהחשבון הראשון חשוב אותם אחדים וחברם עם אלה |
|- | |- | ||
|Multiply also the units that are in the upper line by the tens that are in the second line. | |Multiply also the units that are in the upper line by the tens that are in the second line. | ||
| Line 945: | Line 1,046: | ||
|- | |- | ||
|If tens are received, meaning of a higher rank than the present rank, keep them. | |If tens are received, meaning of a higher rank than the present rank, keep them. | ||
| − | |style="text-align:right;"|ואם עלו עשרות והטעם מדרגה עליונה מזאת | + | |style="text-align:right;"|ואם עלו עשרות והטעם מדרגה עליונה מזאת תפוש בלבך |
|- | |- | ||
|Multiply also the units that are in the bottom line by the hundreds that are in the upper line. | |Multiply also the units that are in the bottom line by the hundreds that are in the upper line. | ||
| Line 951: | Line 1,052: | ||
|- | |- | ||
|Also the units that are in the upper line by the hundreds that are in the bottom line. | |Also the units that are in the upper line by the hundreds that are in the bottom line. | ||
| − | |style="text-align:right;"|וכן האחדים שבשטה העליונה עם המאות שבשטה השפלה | + | |style="text-align:right;"|‫[וכן האחדים שבשטה העליונה]‫<ref>P1031 om.</ref> עם המאות שבשטה השפלה |
|- | |- | ||
|Also the tens that are in the bottom line by the tens that are in the upper line. | |Also the tens that are in the bottom line by the tens that are in the upper line. | ||
|style="text-align:right;"|גם העשרות שבשטה השפלה עם העשרות שבשטה העליונה | |style="text-align:right;"|גם העשרות שבשטה השפלה עם העשרות שבשטה העליונה | ||
|- | |- | ||
| − | | | + | |The rule: the sum of [the positional value of the] ranks is equal to [the positional value of] the product. |
|style="text-align:right;"|והכלל המדרגות אשר בקבוצן תהיינה שוות הסך | |style="text-align:right;"|והכלל המדרגות אשר בקבוצן תהיינה שוות הסך | ||
|- | |- | ||
| | | | ||
| + | :The first with the third generate the fourth, and so the third with the first, and the second with the second. | ||
|style="text-align:right;"|כי הראשונה עם השלשה יעשו ד' וככה השלישית עם הראשונה והשנית עם השנית | |style="text-align:right;"|כי הראשונה עם השלשה יעשו ד' וככה השלישית עם הראשונה והשנית עם השנית | ||
|- | |- | ||
| | | | ||
| + | :Similarly, the first with the fourth generate the fifth, and so the second with the third. | ||
|style="text-align:right;"|גם הראשונה עם הרביעית יעשו חמשה וככה השנית עם השלישית | |style="text-align:right;"|גם הראשונה עם הרביעית יעשו חמשה וככה השנית עם השלישית | ||
|- | |- | ||
| − | | | + | |Proceed constantly and add to it the number you kept that is in the rank we consider as tens. |
| − | |style="text-align:right;"|וככה תעשה עד אין קץ ואשר יהיה תחבר המספר שהיה במדרגה שחשבנוה עשרות שתפשת בלבך עמם וכתבם למטה והוא הסך המבוקש | + | |style="text-align:right;"|וככה תעשה עד אין קץ ואשר יהיה תחבר המספר שהיה במדרגה שחשבנוה עשרות שתפשת בלבך עמם |
| + | |- | ||
| + | |Write them beneath and it is the required number. | ||
| + | |style="text-align:right;"|וכתבם למטה והוא הסך המבוקש | ||
|- | |- | ||
| | | | ||
| Line 1,012: | Line 1,118: | ||
|- | |- | ||
| | | | ||
| + | ::Since there are no units, we write a zero in the first rank and we keep the 40 in our mind as four. | ||
|style="text-align:right;"|ובעבור שלא נשארו אחדים כתבנו גלגל במדרגה הראשונה והמ' תפשנום בלבנו ארבע | |style="text-align:right;"|ובעבור שלא נשארו אחדים כתבנו גלגל במדרגה הראשונה והמ' תפשנום בלבנו ארבע | ||
|- | |- | ||
| | | | ||
:*We multiply 8 by 3; it is 24. Also 4 by 5, for they are of the same rank; it is 20. We add it to the 24; it is 44. | :*We multiply 8 by 3; it is 24. Also 4 by 5, for they are of the same rank; it is 20. We add it to the 24; it is 44. | ||
| − | |||
|style="text-align:right;"|עוד כפלנו ח' עם ג' והיו כ"ד גם ד' עם ה' כי הם שוה המדרגות והיו כ' חברנום עם הכ"ד והיו מ"ד | |style="text-align:right;"|עוד כפלנו ח' עם ג' והיו כ"ד גם ד' עם ה' כי הם שוה המדרגות והיו כ' חברנום עם הכ"ד והיו מ"ד | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|גם חברנו עמהם הד' שתפשנו בלבנו היו מ"ח וכתבנו למטה במדרגת העשרות ח' והמ' תפשנו בלבנו ד' | + | ::We add to it the 4 we kept in our mind; it is 48. |
| + | ::<math>\scriptstyle{\color{blue}{\left(8\sdot3\right)+\left(4\sdot5\right)+4=24+20=4=44+4=48}}</math> | ||
| + | |style="text-align:right;"|גם חברנו עמהם הד' שתפשנו בלבנו היו מ"ח | ||
| + | |- | ||
| + | | | ||
| + | ::We write 8 below in the rank of tens and we keep the 40 in our mind as four. | ||
| + | |style="text-align:right;"|וכתבנו למטה במדרגת העשרות ח' והמ' תפשנו בלבנו ד‫' | ||
|- | |- | ||
| | | | ||
:*We multiply 8 by 2; it is 16. Also 3 by 5; it is 15. Also 4 by 3; it is 12. For all of them are of the same rank. It is 43. | :*We multiply 8 by 2; it is 16. Also 3 by 5; it is 15. Also 4 by 3; it is 12. For all of them are of the same rank. It is 43. | ||
| − | |||
|style="text-align:right;"|עוד כפלנו ח' עם ב' והיו י"ו גם ג' עם ה' והיו ט"ו גם ד' עם ג' והיו י"ב כי כל אלה שוה המדרגות והם מ"ג | |style="text-align:right;"|עוד כפלנו ח' עם ב' והיו י"ו גם ג' עם ה' והיו ט"ו גם ד' עם ג' והיו י"ב כי כל אלה שוה המדרגות והם מ"ג | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|חברנו עמהם הד' שתפשנו בלבנו והם מ"ז כתבנו הז' במדרגת המאות והד' שהם המ' תפשנו בלבנו | + | ::We add to it the 4 we kept in our mind; it is 47. |
| + | ::<math>\scriptstyle{\color{blue}{\left(8\sdot2\right)+\left(3\sdot5\right)+\left(4\sdot3\right)+4=16+15+12+4=43+4=47}}</math> | ||
| + | |style="text-align:right;"|חברנו עמהם הד' שתפשנו בלבנו והם מ"ז | ||
| + | |- | ||
| + | | | ||
| + | ::We write 7 in the rank of hundreds and we keep the 4, which is 40, in our mind. | ||
| + | |style="text-align:right;"|כתבנו הז' במדרגת המאות והד' שהם המ' תפשנו בלבנו | ||
|- | |- | ||
| | | | ||
| Line 1,036: | Line 1,153: | ||
|- | |- | ||
| | | | ||
| + | ::We write 1 in the rank of thousands and we keep the 20 in our mind as two. | ||
|style="text-align:right;"|כתבנו הא' במדרגת האלפים והכ' תפשנו אותם בלבנו שנים | |style="text-align:right;"|כתבנו הא' במדרגת האלפים והכ' תפשנו אותם בלבנו שנים | ||
|- | |- | ||
| | | | ||
:*We multiply 3 by 2; it is 6. | :*We multiply 3 by 2; it is 6. | ||
| − | |||
|style="text-align:right;"|עוד כפלנו ג' עם ב' והיו ו‫' | |style="text-align:right;"|עוד כפלנו ג' עם ב' והיו ו‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|חברנו עמהם הב' שתפשנו בלבנו והיו ח' | + | ::We add to it the 2 we kept in our mind; it is 8. |
| + | ::<math>\scriptstyle{\color{blue}{\left(3\sdot2\right)+2=6+2=8}}</math> | ||
| + | |style="text-align:right;"|חברנו עמהם הב' שתפשנו בלבנו והיו ח‫' | ||
|- | |- | ||
| | | | ||
| + | ::We write 8 in the rank of tens of thousands. | ||
| + | |style="text-align:right;"|כתבנו הח' במדרגת הרבבות | ||
| + | |- | ||
| + | | | ||
| + | :We know from this that the number resulting from multiplying the bottom line by the upper line is eighty-one thousand, seven hundred and eighty. | ||
|style="text-align:right;"|וידענו מזה שהסך העולה מכפל השטה השפלה על השטה העליונה הם שמנים ואחת אלפים ושבע מאות ושמנים | |style="text-align:right;"|וידענו מזה שהסך העולה מכפל השטה השפלה על השטה העליונה הם שמנים ואחת אלפים ושבע מאות ושמנים | ||
|- | |- | ||
| | | | ||
| − | ===== Shortcuts ===== | + | ===== <span style=color:Green>Shortcuts</span> ===== |
| | | | ||
| Line 1,073: | Line 1,197: | ||
|If you want to multiply a number that does not have a third, you take a third of the closest number to it, square it and do according to the rule, then examine if the number, whose third you took, is less than the required number, sum up the two numbers and add them to the amount; the result is the wanted. | |If you want to multiply a number that does not have a third, you take a third of the closest number to it, square it and do according to the rule, then examine if the number, whose third you took, is less than the required number, sum up the two numbers and add them to the amount; the result is the wanted. | ||
:<math>\scriptstyle{\color{OliveGreen}{\left(a+1\right)^2=\left[\left(\frac{1}{3}\sdot a\right)^2\sdot10\right]-\left(\frac{1}{3}\sdot a\right)^2+a+\left(a+1\right)}}</math> | :<math>\scriptstyle{\color{OliveGreen}{\left(a+1\right)^2=\left[\left(\frac{1}{3}\sdot a\right)^2\sdot10\right]-\left(\frac{1}{3}\sdot a\right)^2+a+\left(a+1\right)}}</math> | ||
| − | |style="text-align:right;"|ואם רצית לכפול מספר שאין לו שלישית אתה לוקח שלישית המספר | + | |style="text-align:right;"|ואם רצית לכפול מספר שאין לו שלישית אתה לוקח שלישית המספר יותר קרוב אליו ותרבע אותו ותעשה כמשפט אחר תעיין ואם המספר אשר לקחת שלישיתו הוא יותר פחות מהמספר המבוקש קבץ שני המספרים והוסיפם על הסך וההוה הוא המבוקש |
|- | |- | ||
| | | | ||
| Line 1,082: | Line 1,206: | ||
| | | | ||
:It does not have a third and the closest number that has a third is nine, which is less than it. We take the square of its third, which is 9. We take its analogous in the next rank, which is 90. We subtract 9 from it; 81 remains. Since the number, whose third we took, is less than the required number, we sum up the two numbers, the 9 with the 10; it is 19. We add it to the 81; it is 100 and this is the square of ten. | :It does not have a third and the closest number that has a third is nine, which is less than it. We take the square of its third, which is 9. We take its analogous in the next rank, which is 90. We subtract 9 from it; 81 remains. Since the number, whose third we took, is less than the required number, we sum up the two numbers, the 9 with the 10; it is 19. We add it to the 81; it is 100 and this is the square of ten. | ||
| − | |style="text-align:right;"|וזה אין לו שלישית והמספר הקרוב אליו אשר יש לו השלישית הוא התשעה והוא פחות ממנו לקחנו מרובע שלישיתם והוא ט' ולקחנו | + | |style="text-align:right;"|‫<ref>31r</ref>וזה אין לו שלישית והמספר הקרוב אליו אשר יש לו השלישית הוא התשעה והוא פחות ממנו לקחנו מרובע שלישיתם והוא ט' ולקחנו דמיון מהמדרגה הבאה והוא צ' גרענו ממנו ט' ונשארו פ"א ומפני שהמספר אשר לקחנו השלישית ממנו הוא פחות מהמספר המבוקש קבצנו שני המספרים הט' עם הי' ונהיו י"ט {{#annot:term|178,1206|kYgo}}הוספנום על{{#annotend:kYgo}} הפ"א ונהיו ק' והוא מרובע העשרה |
|- | |- | ||
|colspan=2| | |colspan=2| | ||
| Line 1,098: | Line 1,222: | ||
| | | | ||
:Since it does not have a third and the closest number that has a third is 12, which is greater than it, we take its third and square it, then we take its analogous in the next rank, which is 160. We subtract 16 from it, which is the square of the third; 144 remains. Since the number, whose third we took, which is 12, is greater than the number, whose square is wanted, which is 11, we sum up both [numbers]; it is 23. We subtract it from 144; 121 remains and this is the required, which is the square of 11. | :Since it does not have a third and the closest number that has a third is 12, which is greater than it, we take its third and square it, then we take its analogous in the next rank, which is 160. We subtract 16 from it, which is the square of the third; 144 remains. Since the number, whose third we took, which is 12, is greater than the number, whose square is wanted, which is 11, we sum up both [numbers]; it is 23. We subtract it from 144; 121 remains and this is the required, which is the square of 11. | ||
| − | |style="text-align:right;"|ומפני שאין לו שלישית והמספר הקרוב אליו שיש לו שלישית הוא הי"ב והוא יותר ממנו לקחנו שלישיתו וכפלנוהו במספר המרובע ולקחנו דמיונו במדרגה הבאה והוא ק"ס גרענו ממנו הי"ו שהוא מרובע השלישית ונשארו קמ"ד ומפני שהמספר אשר לקחנו השלישית ממנו שהוא י"ב הוא יותר מהמספר המבוקש | + | |style="text-align:right;"|ומפני שאין לו שלישית והמספר הקרוב אליו שיש לו שלישית הוא הי"ב והוא יותר ממנו לקחנו שלישיתו וכפלנוהו במספר המרובע ולקחנו דמיונו במדרגה הבאה והוא ק"ס גרענו ממנו הי"ו שהוא מרובע השלישית ונשארו קמ"ד ומפני שהמספר אשר לקחנו השלישית ממנו שהוא י"ב הוא יותר מהמספר המבוקש מרבעו שהוא י"א קבצנו שתיהן ונהיו כ"ג וגרענו אותם מן קמ"ד ונשארו קכ"א וזהו המבוקש שהוא מרובע י"א |
|- | |- | ||
|colspan=2| | |colspan=2| | ||
| Line 1,104: | Line 1,228: | ||
|- | |- | ||
|Said Mordecai: I have found a respectable way of multiplication by the method of fifths: | |Said Mordecai: I have found a respectable way of multiplication by the method of fifths: | ||
| − | |style="text-align:right;"|<big>אמר מרדכי</big> מצאתי דרך נכבד בענין הכפל בדרך החמישיות | + | |style="text-align:right;"|‫<big>[אמר מרדכי]</big>‫<ref>P1031 om.</ref> מצאתי דרך נכבד בענין הכפל בדרך החמישיות |
|- | |- | ||
|That is when you wish to multiply a number by itself, take its fifth, multiply it by itself, raise it to the next rank, then multiply it by two and a half and this is the required. | |That is when you wish to multiply a number by itself, take its fifth, multiply it by itself, raise it to the next rank, then multiply it by two and a half and this is the required. | ||
:<math>\scriptstyle{\color{OliveGreen}{a^2=\left(\frac{1}{5}\sdot a\right)^2\sdot10\sdot\left(2+\frac{1}{2}\right)}}</math> | :<math>\scriptstyle{\color{OliveGreen}{a^2=\left(\frac{1}{5}\sdot a\right)^2\sdot10\sdot\left(2+\frac{1}{2}\right)}}</math> | ||
| − | |style="text-align:right;"|והוא כי כאשר תרצה לכפול מספר על עצמו תקח חמישיתו ותכפלהו על עצמו ותעלהו ב{{#annot:term|203,1316|Q4tG}}מעלה{{#annotend:Q4tG}} | + | |style="text-align:right;"|והוא כי כאשר תרצה לכפול מספר על עצמו תקח חמישיתו ותכפלהו על עצמו ותעלהו ב{{#annot:term|203,1316|Q4tG}}מעלה{{#annotend:Q4tG}} הבא הסמוכה לה אחר תכפול אותה על שנים וחצי והוא המבוקש |
|- | |- | ||
| | | | ||
| Line 1,117: | Line 1,241: | ||
| | | | ||
:Its fifth is 12. We multiply it by itself; it becomes 144. We raise it to the next rank; it becomes one thousand, four hundred and forty. We multiply it by 2 and a half; it becomes 3 thousand and 600 and this is the required. | :Its fifth is 12. We multiply it by itself; it becomes 144. We raise it to the next rank; it becomes one thousand, four hundred and forty. We multiply it by 2 and a half; it becomes 3 thousand and 600 and this is the required. | ||
| − | |style="text-align:right;"|וחמישיתו י"ב כפלנום על עצמם נהיו קמ"ד העלנו אותם במדרגה הבאה הסמוכה להם | + | |style="text-align:right;"|וחמישיתו י"ב כפלנום על עצמם נהיו קמ"ד העלנו אותם במדרגה הבאה הסמוכה להם נהיו אלף וארבע מאות וארבעים כפלנום על ב' וחצי ונהיו ג' אלפים ת"ר וזהו המבוקש |
|- | |- | ||
|colspan=2| | |colspan=2| | ||
| Line 1,123: | Line 1,247: | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:50²|857|l9hv}} | + | *{{#annot:50²|857|l9hv}}Example: we wish to multiply fifty by fifty. |
:<math>\scriptstyle50^2</math> | :<math>\scriptstyle50^2</math> | ||
| − | |style="text-align:right;"|<big>דמיון | + | |style="text-align:right;"|<big>דמיון</big> רצינו לכפול חמשים על חמשים{{#annotend:l9hv}} |
|- | |- | ||
| | | | ||
| Line 1,135: | Line 1,259: | ||
|- | |- | ||
|If you want to multiply a number by itself, but it does not have a fifth. Examine the closest number to it that has a fifth, whether preceding it or following it. The difference never exceeds over two. | |If you want to multiply a number by itself, but it does not have a fifth. Examine the closest number to it that has a fifth, whether preceding it or following it. The difference never exceeds over two. | ||
| − | |style="text-align:right;"|ואם רצית לכפול מספר על עצמו ואין לו חמישית תעיין המספר הקרוב אליו שיש לו חמישית אם מלפניו | + | |style="text-align:right;"|ואם רצית לכפול מספר על עצמו ואין לו חמישית תעיין המספר הקרוב אליו שיש לו חמישית אם מלפניו או מלאחריו ולא יעבור ההפרש לעולם על שנים |
|- | |- | ||
|If the closest number to your number that has a fifth precedes your number by one, calculate it by the method I have mentioned first regarding the number that has a fifth, then sum up your number with the number that has a fifth, add it to the amount and this is the required. | |If the closest number to your number that has a fifth precedes your number by one, calculate it by the method I have mentioned first regarding the number that has a fifth, then sum up your number with the number that has a fifth, add it to the amount and this is the required. | ||
:<math>\scriptstyle{\color{OliveGreen}{\left(a+1\right)^2=\left(\frac{1}{5}\sdot a\right)^2\sdot10\sdot\left(2+\frac{1}{2}\right)+a+\left(a+1\right)}}</math> | :<math>\scriptstyle{\color{OliveGreen}{\left(a+1\right)^2=\left(\frac{1}{5}\sdot a\right)^2\sdot10\sdot\left(2+\frac{1}{2}\right)+a+\left(a+1\right)}}</math> | ||
| − | |style="text-align:right;"|ואם היה המספר | + | |style="text-align:right;"|ואם היה המספר שיש לו חמישית הקרוב אל מספרך [קודם ממספרך]‫<ref>P1031 om.</ref> באחד תחשבהו בדרך שהזכרתי ראשונה על המספר שיש לו חמישית אחר תחבר מספרך עם המספר שיש לו חמישית ותוסיפהו על הסך והוא המבוקש |
|- | |- | ||
|If the difference is two, multiply your number, after you sum it up with the number that has a fifth, by two, then add it to the amount and this is the required. | |If the difference is two, multiply your number, after you sum it up with the number that has a fifth, by two, then add it to the amount and this is the required. | ||
| Line 1,146: | Line 1,270: | ||
|- | |- | ||
|If the number that has a fifth follows your number, calculate your it using the number that has a fifth, then add your number to the number once, if the difference is one, or multiply it by 2 after you sum [them] up, if the difference is two. Subtract [the sum] from the amount and the remainder is the required. | |If the number that has a fifth follows your number, calculate your it using the number that has a fifth, then add your number to the number once, if the difference is one, or multiply it by 2 after you sum [them] up, if the difference is two. Subtract [the sum] from the amount and the remainder is the required. | ||
| − | |style="text-align:right;"|אמנם אם המספר שיש לו | + | |style="text-align:right;"|אמנם אם המספר שיש לו חמישית הוא אחר מספרך תחשוב חשבונך על המספר שיש לו החמישית אחר תחבר מספרך עם המספר פעם אחת אם ההפרש אחד או תכפול אותו עם ב' אחר שחברתו אם ההפרש שנים ותגרעהו מהסך והנשאר הוא המבוקש |
|- | |- | ||
| | | | ||
| Line 1,155: | Line 1,279: | ||
| | | | ||
:This number does not have a fifth. The closest number to it that has a fifth is ten. | :This number does not have a fifth. The closest number to it that has a fifth is ten. | ||
| − | |style="text-align:right;"|והנה זה המספר אין לו | + | |style="text-align:right;"|והנה זה המספר אין לו חמישית והמספר הקרוב אליו אשר יש לו חמישית הוא העשרה |
|- | |- | ||
| | | | ||
:We multiply it by the method of the fifths; the result is 100. Then, we sum up the 10 that has a fifth with the 11, which is our number, once, since the difference between our number and 10 is one; the sum is 21. We add it to the 100; it is 121 and this is the required. | :We multiply it by the method of the fifths; the result is 100. Then, we sum up the 10 that has a fifth with the 11, which is our number, once, since the difference between our number and 10 is one; the sum is 21. We add it to the 100; it is 121 and this is the required. | ||
:<math>\scriptstyle{\color{blue}{11^2=10^2+\left(10+11\right)=100+21=121}}</math> | :<math>\scriptstyle{\color{blue}{11^2=10^2+\left(10+11\right)=100+21=121}}</math> | ||
| − | |style="text-align:right;"|וכפלנו אותו בדרך החמישיות ועלו ק' אחר חברנו הי' שיש לו החמישית עם הי"א שהוא | + | |style="text-align:right;"|וכפלנו אותו בדרך החמישיות ועלו ק' אחר חברנו הי' שיש לו החמישית עם הי"א ‫<ref>31v</ref>שהוא מספרנו פעם אחת מפני שההפרש בין מספרנו ובין י' הוא אחד ונהיו כ"א חברנו עם הק' ונהיו קכ"א וזהו המבוקש |
|- | |- | ||
|Another example of a number, such that the difference is two: | |Another example of a number, such that the difference is two: | ||
| Line 1,172: | Line 1,296: | ||
| | | | ||
:This number does not have a fifth. The closest number to it that has a fifth is ten. | :This number does not have a fifth. The closest number to it that has a fifth is ten. | ||
| − | |style="text-align:right;"|והנה זה המספר אין לו חמישית והמספר הקרוב לו בעל | + | |style="text-align:right;"|והנה זה המספר אין לו חמישית והמספר הקרוב לו בעל החמישית הוא העשירי‫' |
|- | |- | ||
| | | | ||
| Line 1,180: | Line 1,304: | ||
|- | |- | ||
|Other examples of numbers, such that the closest number to them that has a fifth comes after them, because in the examples we gave it precedes them: | |Other examples of numbers, such that the closest number to them that has a fifth comes after them, because in the examples we gave it precedes them: | ||
| − | |style="text-align:right;"|דמיון אחר במספרים אשר המספר שיש לו | + | |style="text-align:right;"|דמיון אחר במספרים אשר המספר שיש לו חמישי' הקרוב אליו הוא אחריהם כי הדמיונים אשר עשינו היה בהיותו לפניהם |
|- | |- | ||
| | | | ||
| Line 1,194: | Line 1,318: | ||
:We multiply it by the method of the fifths; the result is 225. Then, we sum up this number that has a fifth with our number, which is 14, once, since the difference is one; it is 29. We subtract it from the amount, since the number that has a fifth follows our number; 196 remains and this is the required. | :We multiply it by the method of the fifths; the result is 225. Then, we sum up this number that has a fifth with our number, which is 14, once, since the difference is one; it is 29. We subtract it from the amount, since the number that has a fifth follows our number; 196 remains and this is the required. | ||
:<math>\scriptstyle{\color{blue}{14^2=15^2-\left(14+15\right)=225-29=196}}</math> | :<math>\scriptstyle{\color{blue}{14^2=15^2-\left(14+15\right)=225-29=196}}</math> | ||
| − | |style="text-align:right;"|כפלנום בדרך החמישיות ועלה רכ"ה אחר חברנו את המספר הזה שיש לו חמישית עם | + | |style="text-align:right;"|כפלנום בדרך החמישיות ועלה רכ"ה אחר חברנו את המספר הזה שיש לו חמישית עם מספרנו שהוא י"ד פעם אחת מפני שההפרש אחד והיה כ"ט גרענום מן הסך מפני כי המספר אשר יש לו החמישית היה אחרי מספרנו ונשארו קצ"ו וזהו המבוקש |
|- | |- | ||
|Example of a number, such that the difference between it and the [number] that has a fifth is two: | |Example of a number, such that the difference between it and the [number] that has a fifth is two: | ||
| − | |style="text-align:right;"|דמיון במספר כזה שההפרש | + | |style="text-align:right;"|דמיון במספר כזה שההפרש בינו ובין אשר יש לו החמישית שנים |
|- | |- | ||
| | | | ||
| Line 1,206: | Line 1,330: | ||
| | | | ||
:This number does not have a fifth. The closest number to it that has a fifth is fifteen. | :This number does not have a fifth. The closest number to it that has a fifth is fifteen. | ||
| − | |style="text-align:right;"|וזה המספר אין לו חמישית והמספר הקרוב | + | |style="text-align:right;"|וזה המספר אין לו חמישית והמספר הקרוב שיש לו החמישית הוא החמשה עשר |
|- | |- | ||
| | | | ||
:We multiply it by the method of the fifths; it is 225. Then, we sum up this number that has a fifth with 13, which is our number; it is 28. We multiply it by 2, since the difference between the two numbers is 2; it is 56. We subtract it from 225, since the number that has a fifth follows our number; 169 remains and this is the required. | :We multiply it by the method of the fifths; it is 225. Then, we sum up this number that has a fifth with 13, which is our number; it is 28. We multiply it by 2, since the difference between the two numbers is 2; it is 56. We subtract it from 225, since the number that has a fifth follows our number; 169 remains and this is the required. | ||
:<math>\scriptstyle{\color{blue}{13^2=15^2-2\sdot\left(15+13\right)=225-\left(2\sdot28\right)=225-56=169}}</math> | :<math>\scriptstyle{\color{blue}{13^2=15^2-2\sdot\left(15+13\right)=225-\left(2\sdot28\right)=225-56=169}}</math> | ||
| − | |style="text-align:right;"|כפלנוהו בדרך החמישיות והיה רכ"ה אחר חברנו זה המספר שיש לו החמישית עם י"ג שהוא מספרנו ונהיו כ"ח כפלנום על ב' מפני שההפרש | + | |style="text-align:right;"|כפלנוהו בדרך החמישיות והיה רכ"ה אחר חברנו זה המספר שיש לו החמישית עם י"ג שהוא מספרנו ונהיו כ"ח כפלנום על ב' מפני שההפרש בין שני המספרים ב' ונהיו נ"ו גרענום מן רכ"ה מפני שהמספר שיש לו החמישית הוא אחרי מספרנו ונשארו קס"ט וזהו המבוקש |
|- | |- | ||
|If you want to multiply a number by a number that are far from the kelal by the same amount, one smaller than it and the other exceeding it, you can multiply the kelal by itself, then square the excess and subtract it from the product of the kelal; the result is the required. | |If you want to multiply a number by a number that are far from the kelal by the same amount, one smaller than it and the other exceeding it, you can multiply the kelal by itself, then square the excess and subtract it from the product of the kelal; the result is the required. | ||
| − | |style="text-align:right;"|ואם תרצה לכפול מספר על מספר שהם רחוקים מהכלל בשווי האחד חסר ממנו והאחר מוסיף עליו אתה יכול לכפול הכלל על עצמו אחר תרבע המספר היתר והחסר ותגרעהו מחשבון הכלל וההוה הוא המבוקש | + | |style="text-align:right;"|ואם תרצה לכפול מספר על מספר שהם רחוקים מהכלל בשווי האחד חסר ממנו והאחר מוסיף עליו אתה יכול לכפול הכלל על עצמו [אחר]‫<ref>P1031 om.</ref> תרבע המספר היתר והחסר ותגרעהו מחשבון הכלל וההוה הוא המבוקש |
|- | |- | ||
| | | | ||
| Line 1,247: | Line 1,371: | ||
| | | | ||
| − | ===== Check ===== | + | ===== <span style=color:Green>Check</span> ===== |
| | | | ||
|- | |- | ||
| − | | | + | |Scales of multiplication by casting out nines [lit. by the Nine way]: |
|style="text-align:right;"|<big>מאזנים</big> על הכפל בדרך הט‫' | |style="text-align:right;"|<big>מאזנים</big> על הכפל בדרך הט‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|תחשוב הטור העליון כולו כאלו הם אחדים | + | :Consider [the ranks of] the whole upper line as if they are units, cast out the nines and keep what remains; it is called the first reserved. |
| + | |style="text-align:right;"|תחשוב הטור העליון כולו כאלו הם אחדים ותשליך אותם ט' ט' והשאר שמרהו וזה יקרא השמור הראשון | ||
|- | |- | ||
| | | | ||
| + | :Do the same with the bottom line; the remainder is called the second reserved. | ||
|style="text-align:right;"|עוד תעשה כן בטור השפל והשאר יקרא השמור השני | |style="text-align:right;"|עוד תעשה כן בטור השפל והשאר יקרא השמור השני | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|תכפול השמור הראשון על השמור השני ואשר יהיה השליכהו ט' ט' והשאר שמרהו וזה יקרא השמור השלישי | + | :Multiply the first reserved by the second reserved, cast out the nines from the result and keep what remains; it is called the third reserved. |
| + | |style="text-align:right;"|תכפול השמור הראשון על השמור השני [ואשר יהיה השליכהו ט' ט']‫<ref>P1031 om.</ref> והשאר שמרהו וזה יקרא השמור השלישי | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|אחר תעיין הטור השלישי השפל אשר יקרא הסך ותחשבהו כאלו הם אחדים והשליכם ט' ט' והשאר עיין ואם הוא שוה לשמור השלישי אפשר שחשבונך יהיה אמת ואם לאו דע בודאי שטעית | + | :Examine the third bottom line, which is called "the product": consider [its ranks] as if they are units and cast out the nines. Then, look if the remainder is equal to the third reserved, your calculation can be correct. If not, know that you were wrong for sure. |
| + | |style="text-align:right;"|אחר תעיין הטור השלישי השפל אשר יקרא הסך ותחשבהו כאלו הם אחדים והשליכם ט' ט' ‫<ref>32r</ref>והשאר עיין ואם הוא שוה לשמור השלישי אפשר שחשבונך יהיה אמת ואם לאו דע בודאי שטעית | ||
|- | |- | ||
| − | | | + | |Scales by casting out sevens [lit. by the Seven way]: |
|style="text-align:right;"|<big>מאזנים</big> בדרך השבעה | |style="text-align:right;"|<big>מאזנים</big> בדרך השבעה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|עיין מספרי הטור הראשון ותתחיל מהיותר כולל ותחשבהו עשרות ואשר בצדו אחדים והשליכם ז' ז' ואשר ישאר | + | :Examine the digits of the first line: start from the highest rank and consider it as tens and what is next to it as units. Cast out the sevens. Consider what remains as tens and what is next to it as units. Cast out the sevens. Do so until you reach the rank of units and keep what remains; it is called the first reserved. |
| + | |style="text-align:right;"|עיין מספרי הטור הראשון ותתחיל מהיותר כולל ותחשבהו עשרות ואשר בצדו אחדים והשליכם ז' ז' ואשר ישאר עוד תחשבהו עשרות ואשר בצדו אחדים והשליכם ז' ז' וכן תעשה [עד שתגיע]‫<ref>P1031 om.</ref> למעלת האחדים ואשר ישאר שמרהו וזה יקרא השמור הראשון | ||
|- | |- | ||
| | | | ||
| + | :Take the digits of the second line and do the same; the remainder is called the second reserved. | ||
|style="text-align:right;"|עוד תקח מספרי הטור השני ועשה כמשפט הזה ואשר ישאר יקרא השמור השני | |style="text-align:right;"|עוד תקח מספרי הטור השני ועשה כמשפט הזה ואשר ישאר יקרא השמור השני | ||
|- | |- | ||
| | | | ||
| + | :Multiply the second reserved by the first reserved and cast out the sevens from the result; what remains is called the third reserved. | ||
|style="text-align:right;"|אחר תכפול השמור השני על השמור הראשון ואשר יהיה השליכהו ז' ז' והשאר יקרא השמור השלישי | |style="text-align:right;"|אחר תכפול השמור השני על השמור הראשון ואשר יהיה השליכהו ז' ז' והשאר יקרא השמור השלישי | ||
|- | |- | ||
| | | | ||
| + | :Then, examine the digits of the third bottom line that is called "the product": do the same as with the upper lines and examine the remainder: if it is equal to the third reserved, your calculation can be correct, otherwise, know that you were wrong for sure. | ||
|style="text-align:right;"|אחר כן תעיין מספרי הטור השפל השלישי אשר יקרא הסך ועשהו כמשפטי הטורים העליונים ועיין הנשאר ואם הוא שוה לשמור השלישי אפשר שחשבונך יהיה אמת ואם לאו דע בודאי שטעית | |style="text-align:right;"|אחר כן תעיין מספרי הטור השפל השלישי אשר יקרא הסך ועשהו כמשפטי הטורים העליונים ועיין הנשאר ואם הוא שוה לשמור השלישי אפשר שחשבונך יהיה אמת ואם לאו דע בודאי שטעית | ||
|} | |} | ||
| Line 1,285: | Line 1,417: | ||
| | | | ||
| − | ==== Euclidean Propositions ==== | + | ==== <span style=color:Green>Euclidean Propositions</span> ==== |
| | | | ||
| Line 1,305: | Line 1,437: | ||
|- | |- | ||
| | | | ||
| − | ::We also multiply | + | ::We also multiply 5 by 12; it is 60. |
| − | |style="text-align:right;"|עוד כפלנו | + | |style="text-align:right;"|עוד כפלנו הה' על הי"ב והיו ס‫' |
|- | |- | ||
| | | | ||
| − | ::We also multiply | + | ::We also multiply 4 by 12; it is 48. |
| − | |style="text-align:right;"|עוד כפלנו | + | |style="text-align:right;"|עוד כפלנו הארבעה על הי"ב והיו מ"ח |
|- | |- | ||
| | | | ||
| Line 1,323: | Line 1,455: | ||
| | | | ||
:*{{#annot:10,7+3|250|KF6o}}Example: the number ten; we divide it into 7 and 3. | :*{{#annot:10,7+3|250|KF6o}}Example: the number ten; we divide it into 7 and 3. | ||
| − | |style="text-align:right;"|דמיון המספר עשרה וחלקנוהו על ז' וג‫'{{#annotend:KF6o}} | + | |style="text-align:right;"|<big>דמיון</big> המספר עשרה וחלקנוהו על ז' וג‫'{{#annotend:KF6o}} |
|- | |- | ||
| | | | ||
| Line 1,341: | Line 1,473: | ||
*[[ספר_היסודות_לאקלידס#Elements_II_3|<span style=color:blue>'''Euclid, Elements, Book II, proposition 3'''</span>]]: {{#annot:(a+b)·b=(a·b)+b²|251|Eu5r}}For any number divided into two parts as you wish, the product of the whole number by any of its two parts is equal to the product of the one part by the other plus the square of the part by which you multiplied the whole number. | *[[ספר_היסודות_לאקלידס#Elements_II_3|<span style=color:blue>'''Euclid, Elements, Book II, proposition 3'''</span>]]: {{#annot:(a+b)·b=(a·b)+b²|251|Eu5r}}For any number divided into two parts as you wish, the product of the whole number by any of its two parts is equal to the product of the one part by the other plus the square of the part by which you multiplied the whole number. | ||
:<math>\scriptstyle{\color{OliveGreen}{\left(a+b\right)\sdot b= \left(a\sdot b\right)+b^2}}</math> | :<math>\scriptstyle{\color{OliveGreen}{\left(a+b\right)\sdot b= \left(a\sdot b\right)+b^2}}</math> | ||
| − | |style="text-align:right;"|עוד כל מספר שחלקת אותו | + | |style="text-align:right;"|עוד כל מספר שחלקת אותו בשני חלקים איך שקרה הנה כפל המספר כולו על אחד משני חלקיו איזה שיהיה שוה לכפול החלק האחד על השני ולמרובע החלק משניהם אשר כפלת על כל המספר{{#annotend:Eu5r}} |
|- | |- | ||
| | | | ||
:*{{#annot:10,3+7|251|zmnp}}Example: the number ten, we divide it into two parts - three and seven. | :*{{#annot:10,3+7|251|zmnp}}Example: the number ten, we divide it into two parts - three and seven. | ||
| − | |style="text-align:right;"|דמיון המספר עשרה חלקנוהו לשני חלקים על שלשה ושבעה{{#annotend:zmnp}} | + | |style="text-align:right;"|<big>דמיון</big> המספר עשרה חלקנוהו לשני חלקים על שלשה ושבעה{{#annotend:zmnp}} |
|- | |- | ||
| | | | ||
| Line 1,355: | Line 1,487: | ||
::Likewise if we multiply 7, which is the other part, by ten; it is seventy and it is equal to the product of 3 by 7, which is 21, plus the square of 7, which is 49, and this is the part by which we multiply; together they are seventy. | ::Likewise if we multiply 7, which is the other part, by ten; it is seventy and it is equal to the product of 3 by 7, which is 21, plus the square of 7, which is 49, and this is the part by which we multiply; together they are seventy. | ||
::<math>\scriptstyle{\color{blue}{\left(7+3\right)\sdot7=10\sdot7=70=21+49=\left(3\sdot7\right)+7^2}}</math> | ::<math>\scriptstyle{\color{blue}{\left(7+3\right)\sdot7=10\sdot7=70=21+49=\left(3\sdot7\right)+7^2}}</math> | ||
| − | |style="text-align:right;"|גם ככה אם היינו כופלים הז' שהוא החלק האחד על העשרה היו שבעים וזה שוה לכפל הג' על הז' שהם כ"א ולמרובע הז' שהם מ"ט שהוא החלק אשר כפלנו ושניהם שבעים | + | |style="text-align:right;"|גם ככה אם היינו כופלים הז' שהוא החלק [האחד]‫<ref>P1031 om.</ref> על העשרה היו שבעים וזה שוה לכפל הג' על הז' שהם כ"א ולמרובע הז' שהם מ"ט שהוא החלק אשר כפלנו ושניהם שבעים |
|- | |- | ||
| | | | ||
| Line 1,364: | Line 1,496: | ||
| | | | ||
:*{{#annot:10,3+7|252|hh0J}}Example: the number ten; we divide it into three and seven. | :*{{#annot:10,3+7|252|hh0J}}Example: the number ten; we divide it into three and seven. | ||
| − | |style="text-align:right;"|דמיון המספר עשרה | + | |style="text-align:right;"|<big>דמיון</big> המספר עשרה חלקנוה על שלשה ושבעה{{#annotend:hh0J}} |
|- | |- | ||
| | | | ||
| Line 1,376: | Line 1,508: | ||
*[[ספר_היסודות_לאקלידס#Elements_II_5|<span style=color:blue>'''Euclid, Elements, Book II, proposition 5'''</span>]]: {{#annot:(½(a+b))²=(a·b)+(b-½(a+b))²=(a·b)+(½(a+b)-a)²|253|43lj}}For any number divided into two equal parts and into two unequal parts, [the sum of] the product of one of the unequal parts by the other and the square of the difference between the two parts, i.e. between the equal part [= the half of the whole number] and the unequal [part] is equal to the square of half the [whole] number. | *[[ספר_היסודות_לאקלידס#Elements_II_5|<span style=color:blue>'''Euclid, Elements, Book II, proposition 5'''</span>]]: {{#annot:(½(a+b))²=(a·b)+(b-½(a+b))²=(a·b)+(½(a+b)-a)²|253|43lj}}For any number divided into two equal parts and into two unequal parts, [the sum of] the product of one of the unequal parts by the other and the square of the difference between the two parts, i.e. between the equal part [= the half of the whole number] and the unequal [part] is equal to the square of half the [whole] number. | ||
:<math>\scriptstyle{\color{OliveGreen}{\left(a\sdot b\right)+\left[\left[\frac{1}{2}\sdot\left(a+b\right)\right]-a\right]^2=\left(a\sdot b\right)+\left[b-\left[\frac{1}{2}\sdot\left(a+b\right)\right]\right]^2=\left[\frac{1}{2}\sdot\left(a+b\right)\right]^2}}</math> | :<math>\scriptstyle{\color{OliveGreen}{\left(a\sdot b\right)+\left[\left[\frac{1}{2}\sdot\left(a+b\right)\right]-a\right]^2=\left(a\sdot b\right)+\left[b-\left[\frac{1}{2}\sdot\left(a+b\right)\right]\right]^2=\left[\frac{1}{2}\sdot\left(a+b\right)\right]^2}}</math> | ||
| − | |style="text-align:right;"|עוד כל מספר כאשר תחלקהו לשני חלקים שוים ולשני חלקים בלתי שוים הנה כפל החלק האחד אל חברו מהחלקים הבלתי שוים ומרובע מה שבין שני החלקים ר"ל בין החלק השוה ובלתי שוה שוה למרובע חצי המספר{{#annotend:43lj}} | + | |style="text-align:right;"|עוד כל מספר כאשר תחלקהו לשני חלקים שוים ולשני חלקים בלתי שוים הנה כפל החלק האחד אל חברו מהחלקים הבלתי שוים ומרובע מה שבין שני ‫<ref>32v</ref>החלקים ר"ל בין החלק השוה ובלתי שוה שוה למרובע חצי המספר{{#annotend:43lj}} |
|- | |- | ||
| | | | ||
:*{{#annot:10,3+7|253|zKhJ}}Example: the number ten, we divide it to five and five, which are equal parts, then we divide it also to 7 and 3, which are unequal parts. | :*{{#annot:10,3+7|253|zKhJ}}Example: the number ten, we divide it to five and five, which are equal parts, then we divide it also to 7 and 3, which are unequal parts. | ||
| − | |style="text-align:right;"|דמיון המספר עשרה | + | |style="text-align:right;"|<big>דמיון</big> המספר עשרה חלקנו לחמשה וחמשה שהם חלקים שוים גם חלקנוהו לז' וג' שהם חלקים בלתי שוים{{#annotend:zKhJ}} |
|- | |- | ||
| | | | ||
| Line 1,392: | Line 1,524: | ||
| | | | ||
::The total is 25 and it is equal to the square of 5, which is the square of half the number. | ::The total is 25 and it is equal to the square of 5, which is the square of half the number. | ||
| − | |style="text-align:right;"והכל כ"ה והם שוים למרובע ה' שהוא מרובע חצי המספר | + | |style="text-align:right;"|והכל כ"ה והם שוים למרובע ה' שהוא מרובע חצי המספר |
|- | |- | ||
|colspan=2| | |colspan=2| | ||
::<math>\scriptstyle{\color{blue}{\scriptstyle\left(3\sdot7\right)+\left[\left(\frac{1}{2}\sdot10\right)-3\right]^2=\left(3\sdot7\right)+\left(5-3\right)^2}}</math><br> | ::<math>\scriptstyle{\color{blue}{\scriptstyle\left(3\sdot7\right)+\left[\left(\frac{1}{2}\sdot10\right)-3\right]^2=\left(3\sdot7\right)+\left(5-3\right)^2}}</math><br> | ||
| − | :<math>\scriptstyle{\color{blue}{\left(3\sdot7\right)+\left[7-\left(\frac{1}{2}\sdot10\right)\right]^2=\left(3\sdot7\right)+\left(7-5\right)^2=\left(3\sdot7\right)+2^2=21+4=25=5^2=\left(\frac{1}{2}\sdot10\right)^2}}</math> | + | ::<math>\scriptstyle{\color{blue}{\left(3\sdot7\right)+\left[7-\left(\frac{1}{2}\sdot10\right)\right]^2=\left(3\sdot7\right)+\left(7-5\right)^2=\left(3\sdot7\right)+2^2=21+4=25=5^2=\left(\frac{1}{2}\sdot10\right)^2}}</math> |
|- | |- | ||
| | | | ||
| Line 1,405: | Line 1,537: | ||
| | | | ||
:*{{#annot:10,2|254|yd9c}}Example: the number ten, we divide it into two halves, which are five each, then we add two to the ten, they are 12. | :*{{#annot:10,2|254|yd9c}}Example: the number ten, we divide it into two halves, which are five each, then we add two to the ten, they are 12. | ||
| − | |style="text-align:right;"|דמיון המספר עשרה וחלקנוהו לשני חצאים שהם כל חצי חמשה {{#annot:term|178,1206|GTDu}}הוספנו{{#annotend:GTDu}} על העשרה שנים והיו י"ב{{#annotend:yd9c}} | + | |style="text-align:right;"|<big>דמיון</big> המספר עשרה וחלקנוהו לשני חצאים שהם כל חצי חמשה {{#annot:term|178,1206|GTDu}}הוספנו{{#annotend:GTDu}} על העשרה שנים והיו י"ב{{#annotend:yd9c}} |
|- | |- | ||
| | | | ||
| Line 1,413: | Line 1,545: | ||
| | | | ||
::We add 25 to it, which is the square of 5 that is half the number; it is 49 and this is equal to the square of 7, which is half the number and the addition together. | ::We add 25 to it, which is the square of 5 that is half the number; it is 49 and this is equal to the square of 7, which is half the number and the addition together. | ||
| − | |style="text-align:right;"|חברנו עמהם כ"ה שהם מרובע ה' שהוא חצי המספר והיו מ"ט וזה שוה למרובע ז' שהוא חצי המספר עם התוספת ביחד | + | |style="text-align:right;"|חברנו עמהם כ"ה שהם מרובע ה' [שהוא]‫<ref>P1031 om.</ref> חצי המספר והיו מ"ט וזה שוה למרובע ז' שהוא חצי המספר עם התוספת ביחד |
|- | |- | ||
|colspan=2| | |colspan=2| | ||
| Line 1,421: | Line 1,553: | ||
*[[ספר_היסודות_לאקלידס#Elements_II_7|<span style=color:blue>'''Euclid, Elements, Book II, proposition 7'''</span>]]: {{#annot:(a+b)²+a²=(2·(a+b)·a)+b²|255|hdYZ}}For any number divided into two parts, the sum of the square of the whole number and the square of one of the parts is equal to twice the product of this part by the whole number plus the product of the other part by itself. | *[[ספר_היסודות_לאקלידס#Elements_II_7|<span style=color:blue>'''Euclid, Elements, Book II, proposition 7'''</span>]]: {{#annot:(a+b)²+a²=(2·(a+b)·a)+b²|255|hdYZ}}For any number divided into two parts, the sum of the square of the whole number and the square of one of the parts is equal to twice the product of this part by the whole number plus the product of the other part by itself. | ||
:<math>\scriptstyle{\color{OliveGreen}{\left(a+b\right)^2+a^2=2\sdot\left[\left(a+b\right)\sdot a\right]+b^2}}</math> | :<math>\scriptstyle{\color{OliveGreen}{\left(a+b\right)^2+a^2=2\sdot\left[\left(a+b\right)\sdot a\right]+b^2}}</math> | ||
| − | |style="text-align:right;"|עוד כל מספר | + | |style="text-align:right;"|עוד כל מספר כשתחלקהו בשני חלקים איך שיקרה הנה המרובע ההוה מן המספר כלו והמרובע ההוה מאחד משני חלקים כאשר {{#annot:term|178,1216|tLsc}}התקבצו{{#annotend:tLsc}} שוים לכפל המספר כלו עם החלק הנזכר פעמים והמרובע ההוה מן החלק השני{{#annotend:hdYZ}} |
|- | |- | ||
| | | | ||
:*{{#annot:10,3+7|255|L1Xh}}Example: the number ten, we divide it randomly to seven and three. | :*{{#annot:10,3+7|255|L1Xh}}Example: the number ten, we divide it randomly to seven and three. | ||
| − | |style="text-align:right;"|דמיון המספר עשרה וחלקנוהו איך שקרה על שבעה ועל שלשה{{#annotend:L1Xh}} | + | |style="text-align:right;"|<big>דמיון</big> המספר עשרה וחלקנוהו איך שקרה על שבעה ועל שלשה{{#annotend:L1Xh}} |
|- | |- | ||
| | | | ||
| Line 1,441: | Line 1,573: | ||
| | | | ||
:*{{#annot:10,3+7|256|AJOP}}Example: we have the number ten, we divide it randomly to 3 and 7. | :*{{#annot:10,3+7|256|AJOP}}Example: we have the number ten, we divide it randomly to 3 and 7. | ||
| − | |style="text-align:right;"|דמיון יש לנו מספר | + | |style="text-align:right;"|<big>דמיון</big> יש לנו מספר מנינו העשרה וחלקנוהו איך שהזדמן על ג' ועל ז‫'{{#annotend:AJOP}} |
|- | |- | ||
| | | | ||
| Line 1,455: | Line 1,587: | ||
| | | | ||
:*{{#annot:10,3+7|257|O8o8}}Example: we have the number ten, we divide it into two equal parts, which are 5, and into two unequal parts, which are 3 and 7. | :*{{#annot:10,3+7|257|O8o8}}Example: we have the number ten, we divide it into two equal parts, which are 5, and into two unequal parts, which are 3 and 7. | ||
| − | |style="text-align:right;"|דמיון יש לנו מספר מנינו עשרה וחלקנוהו לשני חלקים שוים על ה' ושני חלקים בלתי שוים על ג' וז‫'{{#annotend:O8o8}} | + | |style="text-align:right;"|<big>דמיון</big> יש לנו מספר מנינו עשרה וחלקנוהו לשני חלקים שוים על ה' ושני חלקים בלתי שוים על ג' וז‫'{{#annotend:O8o8}} |
|- | |- | ||
| | | | ||
::The square of 7, which is 9, and the square of 3, which is 49, are together 58. This is [as the sum of] double the square of 5, which is 25, and it is half the number, plus double the square of 2, which is 4, that is the excess of 7, which is the greater part, over 5, which is the half; together they are 29. | ::The square of 7, which is 9, and the square of 3, which is 49, are together 58. This is [as the sum of] double the square of 5, which is 25, and it is half the number, plus double the square of 2, which is 4, that is the excess of 7, which is the greater part, over 5, which is the half; together they are 29. | ||
| − | |style="text-align:right;"|הנה מרובע ג' שהוא ט' ומרובע ז' שהוא מ"ט שניהם נ"ח הם כפל מרובע ה' שהוא כ"ה והוא מחצית המספר וכפל מרובע ב' שהוא ד' ושניהם כ"ט שהוא התוספת שיש לז' שהוא החלק הגדול על הה' שהוא המחצית | + | |style="text-align:right;"|הנה מרובע ג' שהוא ט' ומרובע ז' שהוא מ"ט שניהם נ"ח הם כפל מרובע ה' שהוא כ"ה [והוא מחצית המספר]‫<ref>P1031 om.</ref> וכפל מרובע ב' שהוא ד' ושניהם כ"ט שהוא התוספת שיש לז' שהוא החלק הגדול על [הה' שהוא]‫<ref>P1031 om.</ref> המחצית |
|- | |- | ||
|colspan=2| | |colspan=2| | ||
| Line 1,467: | Line 1,599: | ||
*[[ספר_היסודות_לאקלידס#Elements_II_10|<span style=color:blue>'''Euclid, Elements, Book II, proposition 10'''</span>]]: {{#annot:(a+b)²+b²=2·((½a)²+((½a)+b)²)|258|w9pW}}If you divide any number into half and add to it another number, [the sum of] the square of the whole number plus the additional [number] and the square of the additional [number] is equal to twice [the sum of] the square of half the number and the square of half the number plus the additional [number] together. | *[[ספר_היסודות_לאקלידס#Elements_II_10|<span style=color:blue>'''Euclid, Elements, Book II, proposition 10'''</span>]]: {{#annot:(a+b)²+b²=2·((½a)²+((½a)+b)²)|258|w9pW}}If you divide any number into half and add to it another number, [the sum of] the square of the whole number plus the additional [number] and the square of the additional [number] is equal to twice [the sum of] the square of half the number and the square of half the number plus the additional [number] together. | ||
:<math>\scriptstyle{\color{OliveGreen}{\left(a+b\right)^2+b^2=2\sdot\left[\left(\frac{1}{2}\sdot a\right)^2+\left[\left(\frac{1}{2}\sdot a\right)+b\right]^2\right]}}</math> | :<math>\scriptstyle{\color{OliveGreen}{\left(a+b\right)^2+b^2=2\sdot\left[\left(\frac{1}{2}\sdot a\right)^2+\left[\left(\frac{1}{2}\sdot a\right)+b\right]^2\right]}}</math> | ||
| − | |style="text-align:right;"|עוד כל מספר שחלקת אותו לשני חציים והוספת עליו מספר אחר הנה מרובע המספר עם התוספת יחד ומרובע התוספת בעצמו הם כפל שני המרובעים שהם מרובע חצי המספר ומרובע חצי המספר עם התוספת יחד כאשר {{#annot:term| | + | |style="text-align:right;"|עוד כל מספר שחלקת אותו לשני חציים והוספת עליו מספר אחר הנה מרובע המספר עם התוספת יחד ומרובע התוספת בעצמו הם כפל שני המרובעים שהם מרובע חצי המספר ומרובע חצי המספר עם התוספת יחד כאשר {{#annot:term|178,2083|Ycx9}}יחוברו{{#annotend:Ycx9}}{{#annotend:w9pW}} |
|- | |- | ||
| | | | ||
:*{{#annot:10,2|258|zIUc}}Example: we have the number ten, we divide it into two halves, which are 5, and add to it another number 2, so it bacomes 12. | :*{{#annot:10,2|258|zIUc}}Example: we have the number ten, we divide it into two halves, which are 5, and add to it another number 2, so it bacomes 12. | ||
| − | |style="text-align:right;"|דמיון יש לנו מספר מניינו עשרה וחלקנוהו לשני חצאים על | + | |style="text-align:right;"|<big>דמיון</big> יש לנו מספר מניינו עשרה וחלקנוהו לשני חצאים על ה' והוספנו עליו ‫<ref>33r</ref>מספר אחר ב' ונהיה י"ב{{#annotend:zIUc}} |
|- | |- | ||
| | | | ||
| Line 1,496: | Line 1,628: | ||
| | | | ||
| − | === Part Three: | + | === Part Three: To Divide a Number by a Number and to Sum a Number with a Number === |
| − | |style="text-align:right;"|<big>החלק השלישי לחלק מספר על מספר ולהוסיף מספר על מספר | + | |style="text-align:right;"|<big>החלק השלישי</big> לחלק מספר על מספר ולהוסיף מספר על מספר |
|- | |- | ||
| | | | ||
| − | ==== Chapter One: Division ==== | + | ==== Chapter One: <span style=color:Green>Division</span> ==== |
| − | |style="text-align:right;"|<big>הפרק | + | |style="width:45%; text-align:right;"|<big>הפרק הראשון</big> |
| + | |- | ||
| + | |Said Mordecai: We have preceded this chapter to the chapter on proportions, since it precedes proportion by nature and simpler than it. | ||
| + | |style="text-align:right;"|<big>אמר מרדכי</big> הקדמנו זה השער משער הערכים להיותו קודם בטבע [מן הערכי' ופשוט ממנו | ||
| + | |- | ||
| + | |It precedes by nature, because we can find in proportions one term of two or three only by multiplication and division, as will be explained in the chapter, in which we will mention them. | ||
| + | |style="text-align:right;"|אם קודם בטבע]‫<ref>P1031 om.</ref> כי לא נוכל למצוא בערכים הגבול האחד מהשנים או מהשלשה אם לא בדרך הכפל והחלוק כאשר יתבאר בשער אשר נזכירם | ||
|- | |- | ||
| − | | | + | |It is simpler than it, since that chapter consists of this [chapter] and of what is distinctive for it, while this chapter is more specific to both. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואם פשוט ממנו להיות השער ההוא מורכב מזה ומהמיוחד לו וזה השער מיוחד בזה משניהם |
| + | |- | ||
| + | |The arithmeticians carry out this division in two ways: | ||
| + | |style="text-align:right;"|והנה החלוקה הזאת עושים אותה בעלי החשבון על שני דרכים | ||
|- | |- | ||
| − | | | + | |The first way is as mentioned by the late R. Abraham Ibn Ezra the wise. |
|style="text-align:right;"|הדרך האחת כאשר הזכירה החכם ר' אברהם ן' עזרא ז"ל | |style="text-align:right;"|הדרך האחת כאשר הזכירה החכם ר' אברהם ן' עזרא ז"ל | ||
|- | |- | ||
| − | | | + | |The second way is called by the Gentiles "galea" [galley] in their language and its meaning is a boat. |
| − | |style="text-align:right;"|והדרך השנית היא אשר | + | |style="text-align:right;"|והדרך השנית היא אשר קורין אותה הלועזים גליאה בלשו' ופירושו דוגית |
|- | |- | ||
| − | | | + | |I will mention both ways here. |
|style="text-align:right;"|ואני אזכיר הנה גם שתי הדרכים | |style="text-align:right;"|ואני אזכיר הנה גם שתי הדרכים | ||
|- | |- | ||
| − | | | + | |The first way is when you want to divide a number by a number, whether one by many, many by one, or many by many. |
| − | |style="text-align:right;"|הדרך | + | |style="text-align:right;"|<big>הדרך הראשון</big> הוא כאשר תרצה לחלק מספר על מספר בין שיהיה אחד על רבים או רבים על אחד או רבים על רבים |
| + | |- | ||
| + | |First, write the number that you want to divide. | ||
| + | |style="text-align:right;"|תכתוב המספר אשר רצית לחלקו ראשונה | ||
|- | |- | ||
| − | | | + | |In integers, the dividend must always be greater than the divisor. |
| − | |style="text-align:right;"|וראוי שיהיה לעולם המספר המתחלק יותר מהמחולק עליו | + | |style="text-align:right;"|וראוי שיהיה לעולם [המספר המתחלק]‫<ref>P1031 om.</ref> יותר מהמחולק עליו בשלמים |
|- | |- | ||
| − | | | + | |Then, write the number, by which you want to divide it, in the bottom line, and leave a space between the upper line and the bottom line, in order to write between them the result of the division. |
| − | |style="text-align:right;"|אחר תכתוב המספר אשר תרצה לחלק עליו בטור השפל וריוח תשים בין העליון והשפל כדי שתשים ביניהם העולה בחלוק | + | |style="text-align:right;"|אחר תכתוב המספר אשר תרצה לחלק עליו בטור השפל [וריוח תשים בין העליון והשפל]‫<ref>P1031 om.</ref> כדי שתשים ביניהם העולה בחלוק |
|- | |- | ||
| − | | | + | |Beware that you write the bottom number with its ranks corresponding to the ranks of the upper line, each facing its corresponding. |
|style="text-align:right;"|והשמר שתכתוב המספר השפל במדרגותיו כנגד מדרגות הטור העליון כל אחד כנגד הנכחי לו | |style="text-align:right;"|והשמר שתכתוב המספר השפל במדרגותיו כנגד מדרגות הטור העליון כל אחד כנגד הנכחי לו | ||
|- | |- | ||
| − | | | + | |Start dividing from the highest rank. Consider it as units and divide it by the highest rank of the bottom line. |
| − | |style="text-align:right;"|אחר תתחיל לחלק מהכלל היותר גדול ותחשבהו כאלו הם | + | |style="text-align:right;"|אחר תתחיל לחלק מהכלל היותר גדול ותחשבהו כאלו הם אחדים ותחלקהו על הכלל היותר גדול שבטור השפל |
| + | |- | ||
| + | |If the highest rank of the upper line, when you consider it as units, is less than the highest rank of the bottom line, shift it back, so it will be greater than the highest rank of the bottom line and divide by it. | ||
| + | |style="text-align:right;"|ואם הכלל היותר גדול שבטור העליון כשתחשבהו במספר האחדים יהיה יותר מעט מהכלל שבטור השפל החזירהו אחורנית ואז יהיה יותר גדול מהכלל שבטור השפל ותחלק עליו | ||
| + | |- | ||
| + | |See, if the number by which you divide is in the first rank, write the result of division in the last rank; if it is in the second rank, write the result of division in the second rank backwards, i.e. the second from the last; and so on in that order. | ||
| + | |style="text-align:right;"|וראה ואם המספר אשר תחלק עליו הוא מהמדרגה הראשונה כתוב העולה בחלוק במדרגה האחרונה ואם הוא במדרגת השנית כתוב העולה בחלוק במדרגה השנית אחורנית ר"ל השנית לאחרונה וכן על זה הסדר | ||
| + | |- | ||
| + | |If any number remains in the ranks of the first line that cannot be divided in its rank, shift it back and consider it as ten, i.e. any of the remainders that cannot be divided, and add it to the closest lower rank, then divide it. | ||
| + | |style="text-align:right;"|ואם ישאר שום מספר בכללי הטור הראשון אשר לא יכול להתחלק במדרגתו השיבהו אחורנית וחשבהו עשרה ר"ל כל אחד מהנשארים אשר לא יכול להתחלק וחברהו עם המדרגה הקרובה לו השפלה ממנו וחלקהו | ||
|- | |- | ||
| − | | | + | |Do the same if there is a remainder from this rank as well, and so on until you reach the rank of the units. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכן אם ישאר גם מזאת המדרגה ככה תעשה עד שתגיע למדרגת האחדים |
|- | |- | ||
| − | | | + | |You should also know that if the digits by which you divide are numerous, and when you divide the rank of the first line by the rank of the second line nothing remains, you should not give it all the parts, but leave one part, in order to divide it by the rest of the digits in the bottom line and the same for every rank. |
| − | |style="text-align:right;"|וכן ראוי שתדע שאם היו המספרים אשר תחלק עליהם רבים וכשחלקת הכלל שבטור הראשון על הכלל שבטור השני לא נשאר דבר אין ראוי שתתן לו כל החלקים אבל תשאיר חלק אחד כדי שתשיבהו אחורנית ותחלקהו גם על שאר | + | |style="text-align:right;"|וכן ראוי שתדע שאם היו המספרים אשר תחלק עליהם רבים וכשחלקת הכלל שבטור הראשון על הכלל שבטור השני לא נשאר דבר אין ראוי שתתן לו [כל]‫<ref>P1031 om.</ref> החלקים אבל תשאיר חלק אחד כדי שתשיבהו אחורנית ותחלקהו גם על שאר המספר שבטור השפל וכן בכל מדרגה ומדרגה |
|- | |- | ||
| | | | ||
| − | *{{#annot:4215÷14|157|kF9U}}Example: we wish to divide | + | *{{#annot:4215÷14|157|kF9U}}Example: we wish to divide four thousand, two hundred and fifteen by fourteen. |
:<math>\scriptstyle4215\div14</math> | :<math>\scriptstyle4215\div14</math> | ||
| − | |style="text-align:right;"|<big>דמיון</big> רצינו לחלק | + | |style="text-align:right;"|<big>דמיון</big> רצינו לחלק ארבעת אלפים ומאתים ‫<ref>33v</ref>וחמשה עשר על ארבעה עשר{{#annotend:kF9U}} |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :As this diagram: |
| + | |style="text-align:right;"|כזאת הצורה | ||
|- | |- | ||
| | | | ||
| Line 1,581: | Line 1,735: | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|חלקנו הד' שבטור העליון שהוא במדרגת האלפים על האחד שבטור השפל שהוא במדרגת העשרות | + | :We divide the 4 in the upper line, which is in the rank of the thousands, by the one in the bottom line, which is in the rank of the tens. We give it third, in order to be able to divide also by the four units. We write this three in the second rank back from the highest rank, since the one, by which we divide, is in the second rank from the units. |
| + | :<math>\scriptstyle{\color{blue}{4-\left(3\sdot1\right)=4-3=1}}</math> | ||
| + | |style="text-align:right;"|חלקנו הד' שבטור העליון שהוא במדרגת האלפים על האחד שבטור השפל שהוא במדרגת העשרות ונתנו לו שלשה כדי שנוכל לחלק גם על הארבעה האחדים ואלה השלשה כתבנום במדרגה השנית אחורנית תחלתה מהכללים לפי שהאחד אשר חלקנו עליו היה במדרגה השנית מהאחדים | ||
| + | |- | ||
| + | | | ||
| + | :We shift the remaining one back as ten and add it to the two in the rank of hundreds; they become 12. We subtract from it the 4 units three times; nothing remains, since it is related, but [the number] is not gone yet. We write a zero after the 3 in the rank of tens. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(10+2\right)-\left(3\sdot4\right)=12-12=0}}</math> | ||
| + | |style="text-align:right;"|עוד האחד הנשאר השיבונוהו אחורנית לעשרה וחברנוהו עם השני אשר במדרגת המאות ונהיו י"ב חסרנו מהם הד' אחדים שלשה פעמים ולא נשאר דבר להיותו מקושר וכן עדין לא יצא לחוץ כתבנו ספרא אחרי הג' במדרגת העשרות | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|עוד | + | :We divide also the 15 in the upper line by the 14 in the bottom line: we give it one and write it in the rank of units, the first rank, since the dividend is also in the fourth rank from the rank of thousands. One remains undivided. |
| + | :<math>\scriptstyle{\color{blue}{15-\left(1\sdot14\right)=15-14=1}}</math> | ||
| + | |style="text-align:right;"|עוד שבנו לחלק ט"ו שבטור העליון על הי"ד שבטור השפל ונתננו לו אחד וכתבנוהו במדרגת האחדים במדרגה הראשונה מפני שגם המחולקי' הם במדרגה הרביעית ממדרגת האלפים ונשאר אחד בלתי מתחלק | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :We know from this that the quotient is three hundred and one, and one remains undivided. |
| + | |style="text-align:right;"|ומזה ידענו שהחלק הוא שלש מאות ואחד ונשאר גם אחד אשר לא נחלק | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:104034÷114|157|XR8w}}Another example: we wish to divide | + | *{{#annot:104034÷114|157|XR8w}}Another example: we wish to divide one hundred thousand, four thousand, a zero and thirty-four by 114. |
:<math>\scriptstyle104034\div114</math> | :<math>\scriptstyle104034\div114</math> | ||
| − | |style="text-align:right;"|<big>דמיון אחר</big> רצינו לחלק | + | |style="text-align:right;"|<big>דמיון אחר</big> רצינו לחלק מאת אלף וארבעת אלפים וספרא ושלשים וארבעה על קי"ד{{#annotend:XR8w}} |
|- | |- | ||
| | | | ||
| + | :Like this: | ||
|style="text-align:right;"|כזה | |style="text-align:right;"|כזה | ||
|- | |- | ||
| Line 1,614: | Line 1,779: | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :We shift the one in the upper line, which is in the rank of tens of thousand, back as ten in the rank of the thousands. We give the quotient a nine. We subtract from [the ten] nine times one; one remains. We write the nine in the third rank back from the divided rank, because we divide it by one, which is in the third rank from the units. |
| + | :<math>\scriptstyle{\color{blue}{10-\left(1\sdot9\right)=10-9=1}}</math> | ||
| + | |style="text-align:right;"|השיבונוהו האחד שבטור העליון שהוא במדרגת העשר רבבות אל עשרה אחורנית במדרגת הרבבות ונתננו לחלק תשעה הוצאנו תשעה פעמים אחד ממנו נשאר אחד ואלה התשעה כתבנום במדרגה השלישית אחורנית מן הכלל המתחלק כי חלקנום על אחד שהוא במדרגה השלישית מן האחדים | ||
| + | |- | ||
| + | | | ||
| + | :We shift the one that remains in the rank of tens of thousand back to the rank of thousands as ten and add it to the four that is there; they become 14. We subtract from it also nine times one that is in the rank of tens; 5 remains in the rank of thousands. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(10+4\right)-\left(1\sdot9\right)=14-9=5}}</math> | ||
| + | |style="text-align:right;"|והאחד אשר נשאר במדרגת הרבבות השבנוהו אחורנית עשרה במדרגת האלפים וחברנוהו עם הארבעה אשר שם ונהיו י"ד גרענו עוד מהם תשעה פעמים אחד שהוא במדרגת העשרות ונשארו ה' במדרגת האלפים | ||
| + | |- | ||
| + | | | ||
| + | :We subtract from the 0 also 4 times nine that are the units in the bottom line, which it is 36, but it is not enough, so we shift the 4 back to the rank of hundreds as tens; it becomes 40. We subtract from it the 36; 4 remains in the rank of hundreds and 1 in the rank of thousands. | ||
| + | :<math>\scriptstyle{\color{blue}{40-\left(4\sdot9\right)=40-36=4}}</math> | ||
| + | |style="text-align:right;"|עוד גרענו מה0' ד' פעמים תשעה שהם האחדים שבטור השפל והם ל"ו ולא הספיקו השיבונו הד' אחורנית לעשרות שהם במדרגת המאות ונהיו מ' גרענו מהם הל"ו נשארו ד' במדרגת המאות וא' במדרגת האלפים | ||
| + | |- | ||
| + | | | ||
| + | :We divide the 1 that is in the rank of thousands and the 4 that is in the rank of hundreds again by 114 that is in the bottom line. We give the quotient a one and write it in the second rank after the 9. | ||
| + | |style="text-align:right;"|עוד שבנו לחלק הא' שבמדרגת האלפים והד' שבמדרגת המאות על הקי"ד שבטור השפל ונתננו אחד לחלק וכתבנוהו במדרגה השנית אחרי הט‫' | ||
| + | |- | ||
| + | | | ||
| + | :We subtract 1 from the 1 that is in the rank of thousands; nothing remains in the rank of thousands. | ||
| + | :<math>\scriptstyle{\color{blue}{1-1=0}}</math> | ||
| + | |style="text-align:right;"|גרענו א' מהא' אשר במדרגת האלפי' ולא נשאר דבר במדרגת האלפים | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :We subtract also the 1 that is in the rank of tens from the four that is in the rank of hundreds; 3 remains in the rank of hundreds. |
| + | :<math>\scriptstyle{\color{blue}{4-1=3}}</math> | ||
| + | |style="text-align:right;"|עוד גרענו הא' אשר במדרגת העשרות מהארבעה אשר במדרגת המאות נשארו ג' במדרגת המאות | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :We subtract also the 4 units in the bottom line from the 3 that is in the rank of hundreds, but it is not enough, so we shift the 1 back as ten and add it to the 3 that is in the rank of tens; they become 13 and 2 remains in the rank of hundreds. We subtract the four from the 13; 9 remains in the rank of tens. |
| + | :<math>\scriptstyle{\color{blue}{\left(10+3\right)-4=13-4=9}}</math> | ||
| + | |style="text-align:right;"|עוד גרענו הד' האחדים שבטור השפל מהג' שבמדרגת המאות ולא הספיקו ולכן החזרנו הא' אחורנית לעשרה וחברנום עם הג' שבמדרגת העשרות ונהיו י"ג במדרגת המאות נשארו ב' גרענו הארבעה מהי"ג נשארו ט' במדרגת העשרו‫' | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:5214÷108|157|BDX8}}Another example: we wish to divide | + | :We divide again the 2 that remains in the rank of hundreds and the 9 that remains in the rank of tens again by 114. We give the quotient 2 and write it in the rank of units. |
| + | |style="text-align:right;"|עוד שבנו לחלק הב' אשר נשארו במדרגת המאות והט' אשר נשארו במדרגת העשרות על הקי"ד ונתננו ב' לחלק וכתבנוהו במעלת האחדים | ||
| + | |- | ||
| + | | | ||
| + | :We subtract 2 times 1 from the 2; nothing remains. | ||
| + | :<math>\scriptstyle{\color{blue}{2-\left(2\sdot1\right)=0}}</math> | ||
| + | |style="text-align:right;"|והוצאנו ב' פעמים א' מהב' ולא נשאר דבר | ||
| + | |- | ||
| + | | | ||
| + | :We subtract also 2 times 1 from the 9; only 7 remains in the rank of tens. | ||
| + | :<math>\scriptstyle{\color{blue}{9-\left(2\sdot1\right)=7}}</math> | ||
| + | |style="text-align:right;"|עוד הוצאנו ב' פעמים א' [מהט']‫<ref>P1031 מהב'</ref> ולא נשארו במדרגת העשרות רק ז‫' | ||
| + | |- | ||
| + | | | ||
| + | :We subtract also 2 times 4 from the 4 in the upper line, but it is not enough, so we shift 1 back from the 7 that is in the rank of tens as ten and add it to the 4 in the upper line; they become 14. We subtract the 8 from it; 6 remains in the rank of units and 6 in the rank of tens. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(10+4\right)-\left(2\sdot4\right)=14-8=6}}</math> | ||
| + | |style="text-align:right;"|עוד הוצאנו ב' פעמים ד' מהד' שבטור העליון ולא הספיק ולכן החזרנו א' מהז' שהיו על מדרגת העשרו' אחורנית לעשר וחברנום עם הד' שבטור העליון ונהיו י"ד וגרענו מהם הח' ונשארו ו' במדרגת האחדים וו' במדרגת העשרות | ||
| + | |- | ||
| + | | | ||
| + | :We know from this that our number is divided into nine hundred and 12 parts, and 66 remain undivided. | ||
| + | |style="text-align:right;"|וידענו מזה שנחלק מספרנו לתשע מאות וי"ב חלקים ‫<ref>34r</ref>ונשארו גם ס"ו אשר לא נחלקו | ||
| + | |- | ||
| + | |You should know that when you divide the numbers in the upper line by all the numbers in the bottom line, before [the procedure] is complete, if not enough remains there to be divided by the following [digits of the quotient], you should write a zero next to the [digits of the quotient] by which you have divided. Then, when you start dividing by the following [digit], write it next to the zero, as we did in the first example above. | ||
| + | |style="text-align:right;"|וראוי לך לדעת שכאשר תחלק המספרים שבטור העליון על כל המספרים שבטור השפל וקודם שתצא לחוץ הותרו ולא היה לשם השארות שיקשרו עם הבאים אחריהם אז ראוי לך להשים בצד החלקים שחלקת סיפרא ואחרי כן כשתתחיל לחלק הבאים תכתבם בצד הסיפרא כאשר עשינו בדמיון הראשון אשר למעלה מזה | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:5214÷108|157|BDX8}}Another example: we wish to divide five thousand, two hundred, and fourteen by one hundred and eight. | ||
:<math>\scriptstyle5214\div108</math> | :<math>\scriptstyle5214\div108</math> | ||
| − | |style="text-align:right;"|<big>דמיון אחר</big> רצינו לחלק | + | |style="text-align:right;"|<big>דמיון אחר</big> רצינו לחלק חמשת אלפים ומאתים וארבעה עשר על מאה וסיפרא ושמנה{{#annotend:BDX8}} |
|- | |- | ||
| | | | ||
| + | :Like this: | ||
|style="text-align:right;"|כזה | |style="text-align:right;"|כזה | ||
|- | |- | ||
| Line 1,647: | Line 1,864: | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|נתננו לחלק ד' כדי | + | :We give 4 to the quotient and write it in the third rank back, because the one by which we divide is in the third rank from the rank of units. |
| + | |style="text-align:right;"|נתננו לחלק ד' כדי שיספיקו וכתבנוהו במדרגה השלישי' אחורנית כי האחד אשר חלקנום עליו היה במדרגה השלישית ממדרגת האחדים | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :We subtract four times one; 1 remains in the rank of thousands. |
| + | :<math>\scriptstyle{\color{blue}{5-\left(4\sdot1\right)=1}}</math> | ||
| + | |style="text-align:right;"|גרענו ארבעה פעמים אחד ממנו ונשאר א' במדרגת האלפים | ||
|- | |- | ||
| | | | ||
| − | + | :We subtract also 4 times eight, which is 32, from the 1, but it is not enough. So we shift it back as ten; it becomes 12, because we add it to the 2 in the rank of hundreds, but it is still not enough to subtract 32 from it. So we shift 4 from the 12 back as tens and add them to the 1 in the rank of tens; they become 41. 8 remains in the rank of hundreds. We subtract the 32 from 41; 9 remains in the rank of tens. | |
| − | :<math>\ | + | :<math>\scriptstyle{\color{blue}{\left(10+2\right)-4=12-4=8}}</math> |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{\left(40+1\right)-\left(4\sdot8\right)=41-32=9}}</math> |
| + | |style="text-align:right;"|עוד גרענו ד' פעמים שמנה שהם ל"ב מהא' ולא הספיק והשיבונוהו אחורנית לעשרות ונהיו י"ב כי חברנום עם הב' שבמדרגת המאות ועדין אינו מספיק להוציא מהם ל"ב השיבונו אחורנית הד' מהי"ב לעשרות וחברנום עם הא' אשר במדרגת העשרות ונהיו מ"א ונשארו במדרגת המאות ח' וגרענו הל"ב ממ"א ונשארו ט' במדרגת העשרות | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :We divide again the eight by the 1; we give it 8 and write it in the first rank from the units. |
| + | |style="text-align:right;"|עוד שבנו לחלק השמנה על הא' ונתנו לו ח' וכתבנום במדרגה הראשונה מהאחדים | ||
|- | |- | ||
| | | | ||
| + | :We subtract 8 from 8. | ||
| + | |style="text-align:right;"|גרענו ח' מח‫' | ||
| + | |- | ||
| | | | ||
| − | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | + | :We subtract also 8 times 8, which is 64, from the 9 in the rank of tens, but it is not enough. So we shift 6 back to the units; it becomes 60. We add it to the 4 in the upper line; they become 64. We subtract the 64 from it; 3 remains in the rank of tens. |
| + | :<math>\scriptstyle{\color{blue}{9-6=3}}</math> | ||
| + | :<math>\scriptstyle{\color{blue}{\left(60+4\right)-\left(8\sdot8\right)=64-64}}</math> | ||
| + | |style="text-align:right;"|עוד גרענו ח' פעמים ח' שהם ס"ד מהט' שהם במדרגת העשרות ולא הספיקו ולכן השיבונו אחורנית הו' אל האחדים ונהיו ס' וחברנום עם הד' שבטור העליון ונהיו ס"ד וגרענו מהם הס"ד ונשארו ג' במדרגת העשרות | ||
| + | |- | ||
| + | | | ||
| + | :We know from this that the quotient is 48, and thirty remain undivided. | ||
| + | |style="text-align:right;"|וידענו מזה שהחלק הוא מ"ח ונשארו גם שלשים אשר לא נתחלקו | ||
| + | |- | ||
| + | |This is one of the two ways of division. | ||
| + | |style="text-align:right;"|זאת היא הדרך האחת מהשתי דרכים מדרכי החלוקה | ||
| + | |- | ||
| + | !<span style=color:Green>Galea</span> | ||
| + | | | ||
| + | |- | ||
| + | |The second way, which is called in their language "galea" [galley], is the way most traders use. | ||
| + | |style="text-align:right;"|<big>הדרך השנית</big> והיא אשר תקרא בלשונם גליאה היא דרך שרוב הסוחרים משתמשים בה | ||
| + | |- | ||
| + | |It is that you write the number you wish to divide in the upper line and the number, by which you want to divide it, in the bottom line. | ||
| + | |style="text-align:right;"|והוא שתכתוב המספרים אשר תרצה לחלק אותם בטור העליון והמספרים אשר תרצה לחלק עליהם בטור השפל | ||
| + | |- | ||
| + | |Yet, you do not write its ranks corresponding to the upper [ranks], but you write its highest rank, whichever it may be, under the highest rank in the first line and the digit that follows corresponding to the digit that is next to the highest. | ||
| + | |style="text-align:right;"|ולא תכתבם במדרגתם כנגד העליונים אבל איזו מדרגה שיהיו כתב הכולל שלהם תחת הכולל שבטור הראשון והמספר הבא אחריו כנגד המספר אשר בצד הכולל | ||
| + | |- | ||
| + | |Then, you start dividing the highest rank in the upper line by the highest rank in the bottom line, but you do not give it all that you find: | ||
| + | |style="text-align:right;"|אחר תתחיל לחלק הכולל שבטור העליון על הכולל שבטור השפל ולא תתן לו כל מה שתמצא | ||
| + | |- | ||
| + | |If the digits next to it are not enough to be divide by the digits, by which you divide, leave one, or more, as much as you need and see how many times it is found in it, i.e. the highest rank in the other highest rank, after you leave [something] of it, if you leave; write the number of times it is found in it in a special place. | ||
| + | |style="text-align:right;"|ואם אין המספרים אשר בצדו מספיקים לחלקם על המספרים אשר תחלק עליהם תשאיר אחד או יותר לפי מה שתצטרך ותעיין כמה פעמים הוא נכנס בו ר"ל הכולל בכולל אחר שהשארת ממנו אם השארת וכמספר הפעמים אשר יכנס בו כתוב במקום מיוחד המספר ההוא | ||
| + | |- | ||
| + | |Multiply the highest rank of the number, by which you divide, by the number that is written in the special place and subtract [the product] from the highest rank of the dividend; write the remainder above it, if [something] remains and erase the former. | ||
| + | |style="text-align:right;"|ותכה עם המספר הזה הכתוב במקום המיוחד את הכולל מן המספרים אשר תחלק עליהם ותגרעהו מכולל המספר הנחלק והנשאר אם ישאר כתוב אותו למעלה ממנו ותמחוק האחר | ||
| + | |- | ||
| + | |Multiply also the digit that comes after the highest rank of the number, by which you divide, by the number that is written in the special place, subtract [the product] from the digit that comes after the highest rank of the dividend, and proceed according to the rule. | ||
| + | |style="text-align:right;"|עוד תכה עם המספר הכתוב במקום המיוחד את המספר הבא אחר הכולל מהמספר אשר תחלק עליו ותגרעהו מהמספר הבא אחר הכולל מהמספרים הנחלקים ותעשה כמשפט | ||
| + | |- | ||
| + | |Do the same until you divide by all the digits [of the number], by which you divide. | ||
| + | |style="text-align:right;"|וכן תעשה עד שתחלק על כל המספרים ‫<ref>34v</ref>אשר תחלק עליהם | ||
| + | |- | ||
| + | |If the divided digit is not enough to subtract from it, shift back the remaining one, or more, if there is any, as tens, and divide them by the number, by which you divide. Do this until you subtract all the digits, by which you divide. | ||
| + | |style="text-align:right;"|ואם לא הספיק המספר הנחלק לגרוע ממנו תחזיר את האחד הנשאר או היותר אם היו אחורנית לעשרות ותחלקם על המספר אשר תחלק עליהם וכן תעשה עד שתוציא כל המספרים הנחלק עליהם בחלוקה | ||
| + | |- | ||
| + | |Write again the digits, by which you divide: go down one rank, start from there and write [them] in order. | ||
| + | |style="text-align:right;"|עוד תשוב לכתוב המספרים אשר תחלק עליהם ותרד מדרגה אחת ותתחיל משם ותכתוב כסדר | ||
| + | |- | ||
| + | |See how many times the digit in highest rank by which you divide is found in the second digit that comes after the highest rank of the dividend, and write that number, i.e. the number of times, next to the number you wrote in the special place. Multiply by it all the digits, by which you divide, and proceed according to the rule. Subtract the product of the digits, by which you divide, from the first remaining digit of the dividend, erase the written digit and write what remains above it. | ||
| + | |style="text-align:right;"|ותראה כמה פעמים הוא נכנס הכולל מהמספרים אשר תחלק עליהם במספר השני הבא אחר הכולל במספרים הנחלקים והמספר ההוא ר"ל כמות הפעמים כתבהו בצד המספר אשר כתבת במקום המיוחד והכה עמו כל המספרים אשר תחלק עליהם ועשה כמשפט ותוציא הנכפל מהמספרים אשר תחלק עליהם מהראשון שבמספרים המחולקים הנשארים ותמחוק המספר הכתוב ואשר ישאר כתבהו עליו | ||
| + | |- | ||
| + | |Do this until everything is gone. | ||
| + | |style="text-align:right;"|כן תעשה עד שתצא בחוץ | ||
| + | |- | ||
| + | |If, before everything is gone, you have subtract all the digits by which you divide from those digits [of the dividend] and nothing remains, write a zero outside, where you write the quotient, next to the present [digit of] the quotient. Then, divide again the next digits, until everything is gone and write [the following digits of] the quotient next to the zero. | ||
| + | |style="text-align:right;"|ואם בתוך המספר קודם שתצא בחוץ הוצאת כל המספרים אשר חלקת עליהם מהמספרים ההם ולא נשאר דבר כתוב סיפרא בחוץ לשם שאתה כותב החלקים בצד החלק אשר אתה בו עוד תשוב לחלק המספרים הבאים עד שתצא בחוץ והחלקים כתבם בצד הסיפרא | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:4215÷14|157|dIVO}}Example: we wish to divide 4 thousand, two hundred and 15 by 14. | ||
| + | :<math>\scriptstyle4215\div14</math> | ||
| + | |style="text-align:right;"|<big>דמיון</big> רצינו לחלק ד' אלפים ומאתים וט"ו על י"ד{{#annotend:dIVO}} | ||
| + | |- | ||
| + | | | ||
| + | :As this diagram: | ||
| + | |style="text-align:right;"|כזאת הצורה | ||
| + | |- | ||
| + | | | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
|- | |- | ||
|rowspan="2" style="border-bottom: 1px solid black;| | |rowspan="2" style="border-bottom: 1px solid black;| | ||
| Line 1,689: | Line 1,979: | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|כתבנו אותם בטור העליון והי"ד בטור השפל והתחלנו מהמדרגה היותר כוללת ושמנו גם קו אחד יורד בצד המספרים וראינו כמה | + | :We write it in the upper line and the 14 in the bottom line. |
| + | |style="text-align:right;"|כתבנו אותם בטור העליון והי"ד בטור השפל | ||
| + | |- | ||
| + | | | ||
| + | :We start from the highest rank and we draw a descending line next to the numbers. We look how many times 1 is found in 4; we find that it is 4 times, yet we do not give it the whole 4, rather we give it 3, so that it will be enough to divide by the others as well. We write the 3 outside, near the descending line. | ||
| + | |style="text-align:right;"|והתחלנו מהמדרגה היותר כוללת ושמנו גם קו אחד יורד בצד המספרים וראינו כמה פעמים נכנס א' בד' ומצאנו שנכנס ד' פעמים ולא נתננו לו כל הד' אבל נתננו לו ג' כדי שיספיק לחלק גם על השאר והג' כתבנום בחוץ בצד הקו היורד | ||
| + | |- | ||
| + | | | ||
| + | :We subtract the 1 three times from the 4; 1 remains. We erase the 4 and write 1 above in its rank. | ||
| + | :<math>\scriptstyle{\color{blue}{4-\left(1\sdot3\right)=1}}</math> | ||
| + | |style="text-align:right;"|וגרענו הא' ג' פעמים מהד' ונשאר א' ומחקנו הד' וכתבנו למעלה במדרגתו א‫' | ||
| + | |- | ||
| + | | | ||
| + | :We subtract the 4 three times, which is 12, from this remaining one, after we shift it back as ten and add it to the 2; they become 12 and nothing remains. Since nothing remains before we complete, we write a zero next to the 3. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(10+2\right)-\left(4\sdot3\right)=12-12=0}}</math> | ||
| + | |style="text-align:right;"|הוצאנו הד' ג' פעמים שהם י"ב מזה האחד הנותר אחר שהשיבונוהו אחורנית לעשרה וחברנוהו עם הב' ונהיו י"ב ולא נשאר דבר ומפני שלא נשאר דבר קודם שנצא בחוץ כתבנו בצד הג' סיפרא | ||
| + | |- | ||
| + | | | ||
| + | :We divide again the 15 above by the 14: we erase the 14 from its place and write it beneath the 15, because it remains, but it is not related, since it is shifted from its place. | ||
| + | |style="text-align:right;"|עוד שבנו לחלק הט"ו שלמעלה על הי"ד ומחקנו הי"ד ממקומם וכתבנום תחת [הט"ו]‫<ref>P1031: י"א</ref> כי הותירו ואינם קשורים אחר שיצאו ממקומם | ||
| + | |- | ||
| + | | | ||
| + | :We give one to the quotient and write it next to the zero; one part remains undivided, because we subtract the one from the one and erase both, then we subtract the 4 from the 5; 1 remains and we write the one above. | ||
| + | :<math>\scriptstyle{\color{blue}{1-1=0}}</math> | ||
| + | :<math>\scriptstyle{\color{blue}{5-4=1}}</math> | ||
| + | |style="text-align:right;"|ונתננו חלק אחד לאחד וכתבנוהו בצד הסיפרא ונשאר חלק א' בלתי מתחלק כי הוצאנו האחד מן האחד ומחקנו את שתיהם והוצאנו הד' מן הה' ונשאר א' ומחקנו את שתיהן וכתבנו האחד למעלה | ||
| + | |- | ||
| + | | | ||
| + | :We know from this that it is divided into three hundred and one, and 1 remains undivided. | ||
| + | |style="text-align:right;"|וידענו מזה שנחלק לשלש מאות וסיפרא וחלק א' ונשאר גם א' בלתי מתחלק | ||
|- | |- | ||
| | | | ||
*{{#annot:104034÷114|157|WKuz}}Another example: we wish to divide 104034 by 114. | *{{#annot:104034÷114|157|WKuz}}Another example: we wish to divide 104034 by 114. | ||
:<math>\scriptstyle104034\div114</math> | :<math>\scriptstyle104034\div114</math> | ||
| − | |style="text-align:right;"|<big>דמיון אחר</big> רצינו לחלק | + | |style="text-align:right;"|<big>דמיון אחר</big> רצינו לחלק מאת אלף וסיפרא וארבעת אלפים וסיפרא ושלשים וארבעה על קי"ד{{#annotend:WKuz}} |
|- | |- | ||
| | | | ||
| Line 1,731: | Line 2,050: | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|השיבונו | + | :We shift back the one that is in the highest rank of the upper line as ten and we write it in the rank of the tens of thousands, so we write the 114 from there, because it was not enough. |
| + | |style="text-align:right;"|השיבונו האחד שבמספרי הטור העליון הכולל לעשרות וכתבנו אותו במדרגת הרבבות ולכן כתבנו הקי"ד ממנו כי הוא לא הספיק | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|< | + | :We see that the digit by which we divide it is found in it nine times. We write nine outside near the descending line. |
| + | |style="text-align:right;"|וראינו שהמספר אשר נחלק עליו נכנס [בו]‫<ref>P1031 om.</ref> תשעה פעמים וכתבנו התשעה בחוץ בקו היורד בחוץ | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|< | + | :We multiply one by nine; it is 9. We subtract it from the ten in the rank of tens of thousands; 1 remains in the rank of tens of thousands and we erase the one that is in the bottom line. |
| + | :<math>\scriptstyle{\color{blue}{10-\left(1\sdot9\right)=10-9=1}}</math> | ||
| + | |style="text-align:right;"|כפלנו האחד עם התשעה ונהיו ט' גרענו אותם מן העשרה שבמדרגת הרבבות נשאר א' במדרגת הרבבות ומחקנו ‫<ref>35r</ref>האחד שבטור השפל | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|< | + | :We multiply also the 9 by the second 1 in the bottom line; it is 9. We shift the one that is in the rank of tens of thousands back as ten and add it to the four that is in the rank of thousands there; they become 14. We subtract 9 from it; 5 remains. We erase the 1 and the 4 and write 5 above the rank of thousands. |
| − | + | :<math>\scriptstyle{\color{blue}{\left(10+4\right)-\left(9\sdot1\right)=14-9=5}}</math> | |
| − | + | |style="text-align:right;"|[עו' כפלנו הט' עם הא' השני שבטור השפל]‫<ref>P1031 om.</ref> ונהיו ט' החזרנו אחורנית את האחד שבמדרגת הרבבות לעשרו' וחברנוהו עם הארבעה שיש שם במדרגת האלפים [ונהיו י"ד גרענו מהם הט' ונשארו ה']‫<ref>P1031 om.</ref> ומחקנו הא' והד' וכתבנו על מדרגת האלפים ה‫' | |
| − | |||
|- | |- | ||
| | | | ||
| − | + | :We multiply the 4 in the bottom line by 9; it is 36. We shift 4 from the 5 in the rank of thousands to the rank of hundreds as tens; one remains in the rank of thousands. We erase the 5 and write 1 in its place. We shift the 4 back; it becomes forty. We subtract 36 from it; 4 remains in the rank of hundreds. We write it and erase also the 4 in the bottom line. | |
| − | = | + | :<math>\scriptstyle{\color{blue}{5-4=1}}</math> |
| − | + | :<math>\scriptstyle{\color{blue}{40-\left(4\sdot9\right)=40-36=4}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|עוד כפלנו הד' שבטור השפל על הט' ונהיו ל"ו והחזרנו הד' מהה' שבמדרגת האלפי' לעשרות על מדרגת המאות ונשאר אחד במדרגת האלפים ומחקנו הה' וכתבנו במקומו א' והד' אשר החזרנו נהיו ארבעים וגרענו מהם הל"ו ונשארו ד' במדרגת המאות וכתבנום ומחקנו גם הד' בטור השפל |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Since the number is not gone yet, we write the 114 again each of its [digits] one rank before the one in which we first wrote it. |
| + | |style="text-align:right;"|ומפני שהחשבון לא יצא בחוץ עדין שבנו עוד וכתבנו הקי"ד כל א' מהם קודם מדרגה אחת ממה שכתבנום בפעם הראשונה | ||
|- | |- | ||
| | | | ||
| − | + | :We want to divide by it the 1 in the upper line, which is in the rank of thousands, with the numbers that are next to it. We see that it is found in it once. We write 1 outside, next to to the number on the side of the descending line. | |
| − | + | |style="text-align:right;"|ורצינו לחלק הא' אשר בטור העליון שהוא במדרגת האלפים עם המספרים אשר בצדו עליהם וראינו שהם נכנסים בו פעם א' וכתבנו א' בחוץ בצד המספר אשר בצד הקו היורד בחוץ | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :We multiply it by the one in the bottom line; it is 1. We subtract it from the one in the rank of thousands, then we erase it and we erase olse that one. |
| + | :<math>\scriptstyle{\color{blue}{1-\left(1\sdot1\right)=1-1}}</math> | ||
| + | |style="text-align:right;"|כפלנוהו עם האחד שבטור השפל ונהיה א' גרענוהו מן האחד שבמדרגת האלפים ומחקנוהו וגם מחקנו גם זה האחד | ||
|- | |- | ||
| | | | ||
| − | + | :We multiply also the 1 that is outside by the second 1 in the bottom line and subtract it from the 4 in the rank of thousands; three remains. We erase the 4 and write 3 in its place. We erase also the 1. | |
| − | :<math>\scriptstyle\ | + | :<math>\scriptstyle{\color{blue}{4-\left(1\sdot1\right)=4-1=3}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|עוד כפלנו הא' שבחוץ עם הא' השני שבטור השפל וגרענוהו מהד' שבמדרגת המאות ונשארו שלשה ומחקנו הד' וכתבנו במקומם ג' וכן מחקנו גם הא‫' |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :We multiply also the 1 that is outside by the 4 in the bottom line; it is four. We want to subtract it from the 3 that is written in the rank of tens, but it is not enough, so we shift one from the 3 in the rank of hundreds back to the tens. We erase it and write 2 in its place. We add the ten to the 3 in the rank of tens; they become 13. We subtract 4 from it; 9 remains in the rank of tens. We erase the 3 and write 9 instead. We erase also the 4 in the bottom line. |
| + | :<math>\scriptstyle{\color{blue}{3-1=2}}</math> | ||
| + | :<math>\scriptstyle{\color{blue}{\left(10+3\right)-\left(1\sdot4\right)=13-4=9}}</math> | ||
| + | |style="text-align:right;"|עוד כפלנו הא' שבחוץ עם הד' שבטור השפל ונהיו ארבעה רצינו לגרעם מן הג' הכתובים במדרגת העשרות ולא הספיקו ולכן השיבונו מן הג' שבמדרגת המאות אחד אחורנית לעשרות ומחקנום וכתבנו במקומם ב' והעשרות חברנום עם הג' שבמדרגת העשרות ונהיו י"ג גרענו מהם הד' נשארו ט' במדרגת העשרות ומחקנו הג' וכתבנו ט' במקומם וכן מחקנו גם הד' שבטור השפל | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Since the number is not gone yet, we write the 114 again one rank before the rank in which we wrote it on the second time. |
| + | |style="text-align:right;"|עוד מפני שעדיין לא יצא החשבון בחוץ שבנו וכתבנו הקי"ד מדרגה אחת קודם המדרגה שכתבנום בפעם השנית | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :We look how many times it is found in remaining number that is not divided yet. We see that it is found twice. We write 2 outside, on the side of the descending line. |
| + | |style="text-align:right;"|וראינו כמה פעמי' הם נכנסים בחשבון הנשאר אשר לא נתחלק עדין ומצאנו שנכנסים ב' פעמים ולכן כתבנו בחוץ בצד הקו היורד מחוץ ב‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :We multiply it by the 1 in the bottom line; it is 2. We subtract it from the 2 in the rank of thousands; nothing remains, so we erase it and also the one that is in the bottom line. |
| + | :<math>\scriptstyle{\color{blue}{2-\left(2\sdot1\right)=2-2=0}}</math> | ||
| + | |style="text-align:right;"|כפלנו אותם עם הא' שבטור השפל ונהיו ב' גרענום מהב' אשר במדרגת האלפים ולא נשאר דבר ומחקנו אותם וגם הא' שבטור השפל | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|< | + | :We multiply also the 2 by the second 1 in the bottom line; it is 2. We subtract it from the 9 that is written in the rank of tens of the upper line; 7 remains. We erase the 9 and write 7 in its place. We erase also the second 1 in the bottom line. |
| + | :<math>\scriptstyle{\color{blue}{9-\left(2\sdot1\right)=9-2=7}}</math> | ||
| + | |style="text-align:right;"|‫[עוד כפלנו הב' עם הא' השני שבטור השפל]‫<ref>P1031 om.</ref> ונהיו ב' גרענום מהט' שבטור העליון הכתוב במדרגת העשרות ונשארו ז' מחקנו הט' וכתבנו ז' במקומם וכן מחקנו גם הא' השני שבטור השפל | ||
|- | |- | ||
| | | | ||
| − | + | :We multiply also the 2 by the 4 in the bottom line; it is 8. Since there is not enough to subtract it from the 4 that is in the rank of units of the upper line, we shift one from the 7 back as 10. We erase the 7 and write 6 in its place. We add the ten to the 4; they become 14. We subtract 8 from it; 6 remains. We erase the 4 and write 6 instead. We erase also the 4 in the bottom line. | |
| − | :<math>\scriptstyle\ | + | :<math>\scriptstyle{\color{blue}{7-1=6}}</math> |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{\left(10+4\right)-\left(2\sdot4\right)=14-8=6}}</math> |
| + | |style="text-align:right;"|עוד כפלנו הב' עם הד' שבטור השפל ונהיו ח' ומפני כי לא הספיקו לגרעם מן הד' שבטור העליון במדרגת האחדים השיבונו מן הז' אחד אחורנית לי' ומחקנו הז' וכתבנו במקומם ו' והעשרה חברנום עם הד' ונהיו י"ד וגרענו מהם ח' ונשארו ו' ומחקנו את הד' וכתבנו במקומם ו' וכן מחקנו גם הד' שבטור השפל | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :We know from this that it is divided into nine hundred and twelve, and 66 remain undivided. |
| + | |style="text-align:right;"|ומזה ידענו שנחלקו לתשע מאות ושנים עשר חלק ונשארו גם ס"ו אשר לא נתחלקו | ||
|- | |- | ||
| | | | ||
| − | + | ||
| − | :< | + | ===== <span style=color:Green>Check</span> ===== |
| − | |style="text-align:right;"| | + | |
| + | | | ||
| + | |- | ||
| + | |Scales by casting out nines [lit. by the Nine way]: | ||
| + | |style="text-align:right;"|<big>מאזנים על דרך התשעה</big> | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|< | + | :Multiply the scales of the number, by which you multiply, by the scales of the quotient you wrote in the middle according to the first way, or outside, next to the descending line according to the second way. Then, after you multiply them, cast out the nines and keep what remains. |
| + | |style="text-align:right;"|תכפול מאזני המספר אשר [חלקת]‫<ref>P1031 לקחת</ref> עליו עם מאזני החלקים אשר כתבת אותם באמצע לפי הדרך הראשון ולפי הדרך השנית הם אשר כתבת אותם בצד הקו היורד בחוץ ואחר שתכפלם השליכם ט' ט' ואשר ישאר תשמור אותו | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :This is if nothing remains undivided from the number you have divided. |
| + | |style="text-align:right;"|וזה אם לא נשאר מהמספר אשר חלקת אותו שום דבר אשר לא נתחלק | ||
|- | |- | ||
| | | | ||
| − | + | :But, if something remains, take its scales and add it to the number you kept. If they exceed 9, cast out the 9 and the remainder is the real reserved. | |
| − | + | |style="text-align:right;"|אבל אם נשאר קח גם המאזנים שלו וחבר אותם עם המספר ‫<ref>35v</ref>אשר שמרת ואם יעדיפו מהט' תשליך הט' והנשאר הוא השמור האמתי | |
| − | |style="text-align:right;"|< | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Then, examine also the scales of the number you have divided: if it is equal to the reserved, your calculation can be correct, otherwise, know that you were wrong for sure. |
| + | |style="text-align:right;"|אחר תעיין גם אל מאזני המספרים שחלקת אותם ואם הם שוים עם השמור אפשר שחשבונך יהיה אמת ואם לאו דע בודאי שטעית | ||
|- | |- | ||
| − | | | + | |Other scales by casting out sevens [lit. by the Seven way]: |
| − | |style="text-align:right;"|<big> | + | |style="text-align:right;"|<big>מאזנים אחרים</big> על דרך הז‫' |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Consider the ranks of the number, by which you have divided, with those that are next to them as if they are tens and units. Cast out the sevens. Consider what remains also as tens and the number that precedes it as units. Cast out the sevens, [and so on] until you reach the rank of units. What remains is called the scales of the number by which you have divided. |
| + | |style="text-align:right;"|תחשוב כללי המספר אשר חלקת עליהם אלו עם אשר בצדם כאלו הם עשרות ואחדים והשליכם ז' ז' ואשר נשאר תחשבהו עוד לעשרות והמספר אשר בצדו לאחדים והשליכם ז' ז' עד שתגיע למדרגת האחדים ואשר ישאר יקרא מאזני המספר אשר חלקת עליו | ||
|- | |- | ||
| | | | ||
| − | + | :Do the same with the scales of the quotient you wrote in the middle according to the first way, or outside, next to the descending line according to the second way. Cast out the sevens and the remainder is called the scales of the quotient. | |
| − | + | |style="text-align:right;"|עוד כן תעשה במאזני החלקים והם אשר כתבתם באמצע בדרך הראשונה ובחוץ בצד הקו היורד בדרך השנית והשליכם ז' ז' ואשר ישאר יקרא מאזני החלקים | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Then, multiply the scales of the number, by which you multiply, by the scales of the quotient, cast out the nines from the product and keep what remains; it is called the reserved. |
| − | + | |style="text-align:right;"|אחר תכפול מאזני המספר אשר חלקת עליו על מאזני החלקים ואשר יהיה השליכהו ז' ז' ואשר ישאר שמרהו ויקרא השמור | |
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | + | :This is if nothing remains undivided from the number you have divided. | |
| − | + | |style="text-align:right;"|וזה אם לא נשאר במספרים אשר חלקת אותם מספר אשר לא נתחלק | |
| − | |||
| − | |style=" | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :If a number remains undivided, take the scales of that number also this way, cast out the sevens, and add the remainder to the reserved; this is called the real reserved. |
| + | |style="text-align:right;"|אכן אם נשאר מספר אשר לא נתחלק קח מאזני המספר ההוא ג"כ בכזה הדרך והשליכהו ז' ז' והנשאר חברהו עם השמור ואז יקרא השמור באמת | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Then, examine the scales of the number you have divided: this is by extracting it the same way, casting out the sevens, and if what remains is equal to the reserved, your calculation can be correct, otherwise, know that you were wrong for sure. |
| + | |style="text-align:right;"|אחר תעיין אל מאזני המספרים אשר חלקת אותם וזה ג"כ כשתוציאם בזה הדרך והשליכם ז' ז' ואשר ישאר אם הוא שוה לשמור באמת אפשר שיהיה חשבונך אמת ואם לאו דע בודאי שטעית | ||
|- | |- | ||
| − | | | + | |Other scales that are scales of truth: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<big>מאזנים אחרים</big> שהם מאזני צדק |
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | :Multiply the quotient that is in the middle according to the first way, or outside, next to the descending line according to the second way, by the number by which you have divided, if the [product] is equal to the number you have divided - whether all of it is divided, or if not all of it is divided, but it is equal to it after you subtract the undivided number from it - know that your calculation is correct for sure, otherwise, know that you were wrong for sure. |
| + | |style="text-align:right;"|תכפול החלקים אשר באמצע בדרך הראשונה או בצד הקו היורד בחוץ בדרך השנית עם המספר אשר חלקת עליו ואשר יהיה אם הוא שוה עם המספרים אשר חלקת אותם אם נחלקו הכל או אם לא נחלקו הכל אם הוא שוה להם אחר אשר תגרע מהם המספרים אשר לא נחלקו אז תדע בודאי כי חשבונך אמת ואם אין דע בודאי שטעית | ||
|- | |- | ||
| − | | | + | |} |
| − | + | {| | |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | |
| − | + | ==== Chapter Two: To Sum Up a Number with a Number Successively or by another Sequence <span style=color:Green>[= Progression]</span> ==== | |
| + | |||
| + | |style="width:45%; text-align:right;"|<big>הפרק השני</big> להוסיף מספר על מספר כסדרו או על סדר אחר | ||
|- | |- | ||
| − | | | + | |Whoever wants to sum up a number with a number successively, multiplies it by its half plus one half and the result is the required. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{OliveGreen}{\sum_{i=1}^{n} i=n\sdot\left(\frac{1}{2}\sdot n+\frac{1}{2}\right)}}</math> |
| + | |style="text-align:right;"|הרוצה להוסיף מספר על מספר כסדרו יכפול אותו על חציו בתוספת חצי אחד והעולה הוא המבוקש | ||
|- | |- | ||
| | | | ||
| − | *Example: | + | *{{#annot:1-10|669|4op2}}Example: we wish to add the numbers that are from 1 up to 10 one by the one. |
| − | |style="text-align:right;"|<big>דמיון</big> | + | :<math>\scriptstyle\sum_{i=1}^{10} i</math> |
| + | |style="text-align:right;"|<big>דמיון</big> רצינו להוסיף המספרים שהם מא' עד י' [זה על זה]‫<ref>P1031 om.</ref>{{#annotend:4op2}} | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :We multiply 10 by 5; it is 50. We add 5, which is half 10; it is 55 and this is the required. |
| + | :<math>\scriptstyle{\color{blue}{\sum_{i=1}^{10} i=\left[10\sdot\left(\frac{1}{2}\sdot10\right)\right]+\left(\frac{1}{2}\sdot10\right)=\left(10\sdot5\right)+5=50+5=55}}</math> | ||
| + | |style="text-align:right;"|כפלנו הי' בה' ונהיו נ' עוד הוספנו ה' שהוא חצי י' ונהיו נ"ה וזהו המבוקש | ||
|- | |- | ||
| | | | ||
| − | | | + | *{{#annot:1-9|669|vKJf}}Another example for an odd [number of terms]: we wish to know how much is the sum of the numbers from 1 up to 9. |
| − | {| | + | :<math>\scriptstyle\sum_{i=1}^{9} i</math> |
| + | |style="text-align:right;"|<big>דמיון אחר</big> לנפרדים רצינו לדעת כמה תוספת המספרים זה על זה מא' עד ט‫'{{#annotend:vKJf}} | ||
|- | |- | ||
| | | | ||
| − | {|style=" | + | :Its half is 4 and a half. We add to it another half; it is 5. We multiply 9 by 5; it is 45 and this is the required. |
| + | :<math>\scriptstyle{\color{blue}{\sum_{i=1}^{9} i=9\sdot\left[\left(\frac{1}{2}\sdot9\right)+\frac{1}{2}\right]=9\sdot\left[\left(4+\frac{1}{2}\right)+\frac{1}{2}\right]=9\sdot5=45}}</math> | ||
| + | |style="text-align:right;"|והנה חציו ד' וחצי הוספנו על זה עוד חצי אחר ונהיו ה' כפלנו את הט' בה' ונהיו מ"ה וזהו המבוקש | ||
|- | |- | ||
| − | | | + | |All numbers are according to these two examples. |
| − | + | |style="text-align:right;"|ועל אלו שני הדמיונים כל המספרים | |
|- | |- | ||
| − | |} | + | |If you want, square the whole number, add to it its root, then take its half and this is the required. |
| + | :<math>\scriptstyle{\color{OliveGreen}{\sum_{i=1}^{n} i=\frac{1}{2}\sdot\left(n^2+n\right)}}</math> | ||
| + | |style="text-align:right;"|ואם תרצה תרבע כל המספר ותוסיף עליו שרשו וקח מחציתו והוא המבוקש | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|כפלנו | + | *Example: We multiply 10 by itself; it is 100. We add to it its root, which is 10; it is 110. Its half is 55 and this is the required. |
| + | |style="text-align:right;"|<big>דמיון</big> כפלנו הי' על עצמו ונהיו ק' הוספנו עליו שרשו שהוא י' ונהיו ק"י וחציים נ"ה וזהו המבוקש | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{\sum_{i=1}^{10} i=\frac{1}{2}\sdot\left(10^2+\sqrt{10^2}\right)=\frac{1}{2}\sdot\left(100+\sqrt{100}\right)=\frac{1}{2}\sdot\left(100+10\right)=\frac{1}{2}\sdot110=55}}</math> | ||
| + | |- | ||
| + | |Said Mordecai: I have found another very suitable way, which is that you multiply the last required number by the number that follows it, and half the product is the required. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\sum_{i=1}^{n} i=\frac{1}{2}\sdot\left[n\sdot\left(n+1\right)\right]}}</math> | ||
| + | |style="text-align:right;"|<big>אמר מרדכי</big> [מצאתי]‫<ref>P1031 om.</ref> דרך אחרת נכבדת מאד והוא שתכה תכלית המספר המבוקש עם המספר הבא אחריו וחצי ההוה הוא המבוקש | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *{{#annot:1-10|669|QkuV}}Example: we wish to know how much is the sum of the numbers that are from 1 up to 10. |
| + | :<math>\scriptstyle\sum_{i=1}^{10} i</math> | ||
| + | |style="text-align:right;"|<big>דמיון</big> רצינו לדעת כמה תוספת המספרים שהם מא' עד י‫'{{#annotend:QkuV}} | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :We multiply 10 by 11, which is the number that follows it; it is 110. Its half is 55 and this is the required. |
| + | :<math>\scriptstyle{\color{blue}{\sum_{i=1}^{10} i=\frac{1}{2}\sdot\left[10\sdot\left(10+1\right)\right]=\frac{1}{2}\sdot\left(10\sdot11\right)=\frac{1}{2}\sdot110=55}}</math> | ||
| + | |style="text-align:right;"|הכינו הי' עם הי"א שהוא המספר הבא אחריו ונהיו ק"י וחציו נ"ה והוא המבוקש | ||
|- | |- | ||
| | | | ||
| + | *{{#annot:aₙ, 1, 55|669|GzAy}}Question: if a person asks you: I summed numbers and they are 55, how much are the numbers? | ||
| + | :<math>\scriptstyle\sum_{i=1}^{n} i=55</math> | ||
| + | |style="text-align:right;"|‫<ref>36r</ref><big>שאלה</big> אם ישאלך אדם חברתי מספרים והיו נ"ה כמה יהיו המספרים{{#annotend:GzAy}} | ||
| + | |- | ||
| | | | ||
| − | + | :Answer: multiply [the total sum of] the numbers, which is 55, by 2, take the root of the preceding square and see if it is equal to the number that remains [between] the preceding square [and double the sum], then it is [the number of] the numbers. | |
| + | |style="text-align:right;"|<big>תשובה</big> כפול המספרים הנ"ה על ב' וקח שרש המרובע שעבר וראה ואם הוא שוה למספר הנשאר מהמרובע שעבר כמוהם היו מספרים | ||
|- | |- | ||
| | | | ||
| − | {|style=" | + | :In the example: we double the 55; it is 110. The preceding square is 100, its root is 10 and the number left [between] the preceding square [and] double the 55, which is 110, is 10. Hence, the numbers you summed so that they are 55 are 10. |
| + | :<math>\scriptstyle{\color{blue}{2\sdot55-10^2=110-100=10}}</math> | ||
| + | |style="text-align:right;"|המשל כפלנו הנ"ה והיו ק"י והמרובע [שעבר]‫<ref>P1031 om.</ref> ק' ושרשו י' והמספר הנשאר מהמרובע שעבר שהוא ק' עד כפל הנ"ה שהם ק"י הוא י' ואם כן המספרים שחברת והיו נ"ה הם י‫' | ||
|- | |- | ||
| − | | | + | | |
| − | + | *{{#annot:4-10|669|A9Sh}}Question: I summed from 4 up to 10. How much are they? | |
| − | |- | + | :<math>\scriptstyle\sum_{i=4}^{10} i</math> |
| − | |} | + | |style="text-align:right;"|<big>שאלה</big> חברתי מד' עד י' כמה יהיו{{#annotend:A9Sh}} |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Answer: do as the first rule; you find them 55. Then, sum up from 1 to 3; you find them 6. Subtract it from 55; 49 remains and it is the required. |
| + | :<math>\scriptstyle{\color{blue}{\sum_{i=4}^{10} i=\sum_{i=1}^{10} i-\sum_{i=1}^{3} i=55-6=49}}</math> | ||
| + | |style="text-align:right;"|<big>תשובה</big> תעשה כמשפט הראשון ותמצאם נ"ה אחר תחבר מא' עד ג' ותמצאם ו' גרעם מנ"ה ונשארו מ"ט וזהו המבוקש | ||
|- | |- | ||
| − | | | + | |If someone asks you how much are the squares of the numbers up to a certain number successively: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<big>ואם</big> ישאלך אדם כמה הם [מרובעי]‫<ref>P1031 מרביעי</ref> המספרים עד מספר פלוני על הסדר |
|- | |- | ||
| − | | | + | |Answer that he should find the sum of [the numbers themselves], then multiply it by two-thirds of the last number plus one third and the result is the required. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{OliveGreen}{\sum_{i=1}^{n} i^2=\left(\sum_{i=1}^{n} i\right)\sdot\left(\frac{2}{3}\sdot n+\frac{1}{3}\right)}}</math> |
| + | |style="text-align:right;"|תשיב שימצא {{#annot:term|388,1208|TgJj}}חבורם{{#annotend:TgJj}} בפשוטיהם ואחר יכה אותם בשני שלישי תכלית המספר המבוקש עם תוספת שליש אחד ואשר יהיה הוא המבוקש | ||
|- | |- | ||
| | | | ||
| − | | | + | *{{#annot:1-4|672|pOla}}Example: how much are the squares that are summed from 1 up to 4? |
| − | {| | + | :<math>\scriptstyle\sum_{i=1}^{4} i^2</math> |
| + | |style="text-align:right;"|<big>דמיון</big> כמה הם המרובעים המחוברים מא' עד ד‫'{{#annotend:pOla}} | ||
|- | |- | ||
| | | | ||
| − | + | :We multiply [four] by its half plus one half; it is 10. We take two-thirds of four plus one third; its is three. We multiply it by the ten; it is thirty and this is the required. | |
| + | |style="text-align:right;"|כפלנו אותם בחציים עם תוספת חצי אחד ונהיו י' עוד לקחנו שני שליש ארבעה עם תוספת שליש אחר והם שלשה הכינו אותם עם העשרה ונהיו שלשים וזהו המבוקש | ||
|- | |- | ||
| − | | | + | |colspan=2| |
| − | + | :<math>\scriptstyle{\color{blue}{\sum_{i=1}^{4} i^2=\left(\sum_{i=1}^{4} i\right)\sdot\left(\frac{2}{3}\sdot4+\frac{1}{3}\right)=\left[4\sdot\left(\frac{1}{2}\sdot4+\frac{1}{2}\right)\right]\sdot3=10\sdot3=30}}</math> | |
|- | |- | ||
|} | |} | ||
| + | {| | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | |
| − | + | === Part Four: To Relate a Number To a Number === | |
| + | |||
| + | |style="width:45%; text-align:right;"|<big>החלק הרביעי</big> לערוך מספר על מספר | ||
|- | |- | ||
| − | | | + | |In this chapter we will talk about integers because fractions have another way, which we will explain in the next chapter. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|זה השער נדבר בו על שלמים כי לנשברים יש דרך אחרת נבאר אותה בפרק הבא |
|- | |- | ||
| − | | | + | |We say that although [the types of] proportions are ten, the ancients chose only three kinds of proportions that are: arithmetic proportion, geometric proportion and harmonic proportion. We will mention here only the geometric proportion, because it is needed for most of the questions that are asked in arithmetic. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ונאמר שעם היות שהאמצעיים הם עשרה אבל הקדמונים לא בחרו רק שלשת מיני הערכים והם ערכי החשבון וערכי המדות וערכי הנגונים ואנחנו לא נזכיר הנה רק ערכי המדות כי הם צורך לרוב השאלות הנעשות בחשבון |
|- | |- | ||
| − | | | + | |It has already been clarified in geometry that proportion is not possible with less than three terms. Even if the mean term is represents two terms, a proportion occurs with four terms. |
| − | | | + | |style="text-align:right;"|וכבר התבאר בחכמת השעור כי התיחסו' לא תתכן בפחות משלשה גבולים ואם הגבול האמצעי הוא בעד שני גבולים התיחסות תפול בד' גבולים |
| − | |||
|- | |- | ||
| − | | | + | |Therefore, for all the numbers, such that the ratio of the first to the second is as the ratio of the third to the fourth, if you sum the squares of the four of them, it is equal to [the sum of] the square of the first and the fourth summed together and the square of the difference between the second and the third. |
| − | + | |style="text-align:right;"|מפני זה כל המספרים אשר יחס הראשון אל השני כיחס השלישי אל הרביעי אם תחבר מרובע ארבעתם יהיה שוה למרובע הראשון והרביעי מחוברים ומרובע ההפרש שיש בין השני והשלישי | |
| + | |- | ||
| + | |Also, if you sum the square of the second and the third together with the square of the difference between the first and the fourth it is equal to this number. | ||
| + | |style="text-align:right;"|וכן אם תחבר מרובע השני והשלישי יחד עם מרובע ההפרש שיש בין הראשון לרביעי יהיה שוה בחשבון הזה | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{a_1:a_2=a_3:a_4\longrightarrow a_1^2+a_2^2+a_3^2+a_4^2=\left(a_1+a_4\right)^2+\left(a_2-a_3\right)^2=\left(a_2+a_3\right)^2+\left(a_1-a_4\right)^2}}</math> | ||
|- | |- | ||
| − | | | + | |Similarly, when the proportional terms are switched, because, the ratio of the first to the third is as the ratio of the second to the fourth. |
| − | | | + | :<math>\scriptstyle{\color{OliveGreen}{a_1:a_3=a_2:a_4}}</math> |
| + | |style="text-align:right;"|וכן כאשר הומרו היו מתיחסים כי יחס הראשון אל השלישי יהיה כיחס השני אל הרביעי | ||
|- | |- | ||
| − | | | + | |Therefore, from the three knowns we know the fourth unknown. |
| + | |style="text-align:right;"|ומפני זה מהשלשה הידועים נדע הרביעי המוסכל | ||
|- | |- | ||
| − | | | + | |The arithmeticians always tend to write the unknown at the last end, whether it is [one] of the means, or it is the first extreme, provided that one knows how to arrange the others. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכבר נהגו בעלי החשבון לכתוב המוסכל בקצה האחרון לעולם בין שיהיה מהאמצעיים ובין שיהיה הקצה הראשון ובלבד שידע איך יסדר האחרים |
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|<big>דמיון</big> | + | *Example: if one asks: if the 8 yield 4, how much will the 6 yield? |
| + | :<math>\scriptstyle8:4=6:X</math> | ||
| + | |style="text-align:right;"|<big>דמיון</big> אם שאל שואל שאם השמנה ירויחו ד' הו' מה ירויחו | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :The means are the 4 and the 6 and the two extremes are the eight and the zero, like this: |
| + | |style="text-align:right;"|הנה נקראו האמצעיים הד' והו' ושתי הקצוות השמנה והגלגל כזה | ||
|- | |- | ||
| | | | ||
| Line 1,954: | Line 2,341: | ||
{|style="margin-left: auto; margin-right: 0px; border-collapse: collapse;" | {|style="margin-left: auto; margin-right: 0px; border-collapse: collapse;" | ||
|- | |- | ||
| − | |0|| | + | |0||6||4||8 |
|} | |} | ||
|- | |- | ||
| Line 1,960: | Line 2,347: | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|כפלנו האמצעיים ונהיו | + | :We multiply the two means; it is 24. We divide it by the known extreme, which is 8; the quotient is 3. We know from this that 3 should be instead of the zero and this is the required. |
| + | :<math>\scriptstyle{\color{blue}{X=\frac{4\sdot6}{8}=\frac{24}{8}=3}}</math> | ||
| + | |style="text-align:right;"|כפלנו שני האמצעיים ונהיו כ"ד חלקנום על הקצה הידוע שהם הח' ונהיה החלק ג' ומזה ידענו שבמקום הגלגל צריך ג' והוא המבוקש | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *Similarly, if he switches the order and asks: if the 4 yield 8, how much will yield 6? |
| + | :<math>\scriptstyle4:8=X:6</math> | ||
| + | |style="text-align:right;"|וכן אם הפך הסדר ושאל אם ‫<ref>36v</ref>הד' הרויחו אותם הח' מהו אשר ירויחו הו‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Now the two means are the eight and the zero, like this: |
| + | |style="text-align:right;"|ועתה נהיו שני האמצעיים השמנה והגלגל כזה | ||
|- | |- | ||
| | | | ||
| Line 1,975: | Line 2,367: | ||
{|style="margin-left: auto; margin-right: 0px; border-collapse: collapse;" | {|style="margin-left: auto; margin-right: 0px; border-collapse: collapse;" | ||
|- | |- | ||
| − | | | + | |6||0||8||4 |
|} | |} | ||
|- | |- | ||
|} | |} | ||
| + | |- | ||
| + | |When we multiply the two extremes and divide by the known mean, we find the unknown mean. | ||
| + | |style="text-align:right;"|והנה כשנכפול שתי הקצוות ונחלקהו על האמצעי הידוע נמצא האמצעי המוסכל | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|כפלנו | + | :We multiply 4 by 6; it is 24. We divide it by 8; the quotient is 3 and this is the required. |
| − | + | :<math>\scriptstyle{\color{blue}{X=\frac{4\sdot6}{8}=\frac{24}{8}=3}}</math> | |
| − | + | |style="text-align:right;"|כפלנו ד' על ו' ונהיו כ"ד חלקנום על ח' ונהיה החלק ג' וזהו המבוקש | |
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *Likewise, if he turns the question around, he writes the zero first and says: how much will the 6 yield, if the 8 yield 4? |
| + | :<math>\scriptstyle6:X=8:4</math> | ||
| + | |style="text-align:right;"|וכן אם הפך השאלה ושם הגלגל ראשונה ואמר מה ירויחו הו' אם הח' ירויחו ארבעה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :As follows: |
| + | |style="text-align:right;"|כזה | ||
|- | |- | ||
| | | | ||
| Line 1,999: | Line 2,396: | ||
{|style="margin-left: auto; margin-right: 0px; border-collapse: collapse;" | {|style="margin-left: auto; margin-right: 0px; border-collapse: collapse;" | ||
|- | |- | ||
| − | + | |4||8||6||0 | |
|} | |} | ||
|- | |- | ||
|} | |} | ||
| + | |- | ||
| + | |We multiply the known extreme by the mean that is next to the unknown, then divide by the mean that is next to the known extreme and we find the unknown. | ||
| + | |style="text-align:right;"|נכפל הקצה הידוע עם האמצעי אשר בצד המוסכל ונחלקהו על האמצעי אשר בצד הקצה הידוע ונמצא המוסכל | ||
| + | |- | ||
| + | |Even though all of these are the right way, the arithmeticians always make the zero the last extreme, provided that they keep order, so that those who yield the profit are in their place and the profit is in its place. | ||
| + | |style="text-align:right;"|ואע"פ שכל אלה הם דרך נכונה אבל לעולם בעלי החשבון יהפכו הגלגל אל הקצה האחרון בלבד שישמרו הסדר עד שיהיו המרויחים במקומם והריוח במקומו | ||
| + | |- | ||
| + | |Also, if there were measures and dinars, the dinars are in their place and the measures in their place - one should be careful not to replace them, because he would be wrong. | ||
| + | |style="text-align:right;"|וכן אם היו מדות ודינרים שיהיו הדינרים במקומם והמדות במקומם ויזהר שלא יחליף כי יטעה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *{{#annot:wheat|630|IxYr}}Example: one asks: a man sells 7 measures of wheat for 12 pešuṭim. How many measures will he sell for 14 pešuṭim? |
| + | :<math>\scriptstyle7:12=X:14</math> | ||
| + | |style="text-align:right;"|<big>דמיון</big> אם שאל שואל שאדם אחד מוכר חטה ז' מדות בי"ב פשוטים כמה מדות יתן בי"ד פשוטים{{#annotend:IxYr}} | ||
|- | |- | ||
| | | | ||
| − | + | :One should switch the line, provided that he keeps order, and write as follows: if 12 pešuṭim yield 7 measures, how much will 14 pešuṭim yield? | |
| − | = | + | :<math>\scriptstyle12:7=14:X</math> |
| − | + | |style="text-align:right;"|ראוי להפך השטה לבד שישמור הסדר ושיכתוב כך אם י"ב פשוטים נתנו ז' מדות הי"ד פשוטים מה יתנו | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | :Then the zero alway falls at the [last] extreme, in this order: |
| + | |style="text-align:right;"|ואז נופל הגלגל בתכלית האחד ולעולם בזה הסדר | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align: | + | | |
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
|- | |- | ||
| − | | | + | | |
| − | |style=" | + | {|style="margin-left: auto; margin-right: 0px; border-collapse: collapse;" |
| + | |- | ||
| + | |0||14||7||12 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
|- | |- | ||
| − | | | + | |One multiplies the means and divide [the product] by the first extreme, then he deduces the last extreme. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|הוא כופל האמצעיים ומחלק אותם על הקצה הראשון ולומד על הקצה האחרון |
|- | |- | ||
| − | | | + | |When you divide the product by the divisor and it is not reduced, but a number remains undivided, see, if this undivided number counts the number by which it is divided, know that it is its part as many times as it counts it: if it counts it twice, it is its half; if thrice, it is its third; and so on. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכאשר תחלק הכפול על הנחלק עליו ולא יצא בצמצום אבל נשאר חשבון אשר לא נתחלק [תעיין ואם זה החשבון אשר לא נתחלק‫]‫<ref>P1031 om.</ref> הוא תחת כפלי החשבון אשר נתחלק עליו דע כי הוא חלק ממנו לפי הפעמים אשר ישערהו כי אם ישערוהו ב' פעמים הוא מחציתו ואם בג' פעמים הוא שלישיתו וכיוצא בם |
|- | |- | ||
| − | + | |When it does not count it, if you want, extract the part that counts it, then decompose the remainder into fractions, or, if you want, decompose all into fractions, then divide. If there is still a remainder, decompose it into fractions of fractions, until it is reduced, or until something remains that is unperceivable. | |
| − | | | + | |style="text-align:right;"|ואם לא יהיה תחת כפליו אם תרצה תוציא ממנו חלק אשר יהיה תחת כפליו והשאר השיבהו אל פרטים או אם תרצה השב הכל אל הפרטים וחלקהו ואם ישאר עוד השיבהו אל פרטי פרטים עד שיצא בצמצום או עד שישאר דבר שאין חששא בו |
|- | |- | ||
| | | | ||
| − | *Example: | + | *Example: we ask if 12 dinar yield a profit of seven dinar, how much will 14 dinar yield? |
| − | :<math>\ | + | :<math>\scriptstyle12:7=14:X</math> |
| − | |style="text-align:right;"|<big>דמיון</big> | + | |style="text-align:right;"|<big>דמיון</big> שאלנו אם הי"ב דינרים נתנו שבעה דינרים ריוח הי"ד [דינ']‫<ref>P1031 om.</ref> כמה יתנו |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :We write thm as follows: |
| + | |style="text-align:right;"|וכתבנו אותם כך | ||
|- | |- | ||
| | | | ||
| − | |||
| − | |||
| | | | ||
| − | + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | |
| − | |||
| − | |style="text-align: | ||
|- | |- | ||
| | | | ||
| − | |style=" | + | {|style="margin-left: auto; margin-right: 0px; border-collapse: collapse;" |
|- | |- | ||
| − | | | + | |0||14||7||12 |
| − | | | + | |} |
|- | |- | ||
| − | | | + | |} |
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :We multiply the means; it is 98. We divide it by the one extreme, which is 12; the quotient is 8 and 2 remains undivided. Since the 2 are parts of 12 and they count it six times, we know from this that the required is 8 and one sixth. |
| + | :<math>\scriptstyle{\color{blue}{X=\frac{7\sdot14}{12}=\frac{98}{12}=6+\frac{2}{12}=6+\frac{1}{6}}}</math> | ||
| + | |style="text-align:right;"|כפלנו האמצעיים ונהיו צ"ח חלקנום על הקצה האחד שהם י"ב ונהיה החלק הא' ח' ונשארו גם ב' אשר לא נתחלקו ומפני שאלה הב' הם תחת כפלי הי"ב וישערום בששה פעמים ידענו מזה שהמבוקש הוא ח' וששית | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *But, if one asks: if 11 dinar yield a profit of 7 dinar, how much will 14 yield? |
| + | |style="text-align:right;"|אבל אם שאל אם הי"א דינרים נתנו [ז']‫<ref>P1031 י"א</ref> דינרים ריוח הי"ד מה יתנו | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :We write them as follows: |
| + | |style="text-align:right;"|כתבנום כך | ||
|- | |- | ||
| | | | ||
| − | |||
| − | |||
| | | | ||
| − | + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | |
| − | |||
| − | |style="text-align: | ||
|- | |- | ||
| | | | ||
| − | |style=" | + | {|style="margin-left: auto; margin-right: 0px; border-collapse: collapse;" |
|- | |- | ||
| − | | | + | |0||14||7||11 |
| − | | | + | |} |
|- | |- | ||
| − | | | + | |} |
| − | |||
|- | |- | ||
| | | | ||
| − | + | :We multiply the means; it is 98. We divide it by the 11; the quotient is 8 and 10 remains undivided. We decompose it into fractions that are 12 in one dinar; it is 120. We divide it by 11; the quotient is 10 fractions and 10 remains undivided. We decompose it into fractions of fractions that are also 12 in one peruṭa; it is 120. We divide it by 11; the quotient is 10 and 10 remains undivided. Since it is unperceivable, we leave it. So, we know from this that the quotient is 8 dinar, 10 peruṭot of 12 in one dinar, 10 peruṭot of peruṭot of 12 peruṭot, and an unperceivable thing; this is the profit that is received from 14 dinar. | |
| − | :<math>\scriptstyle\ | + | |style="text-align:right;"|כפלנו האמצעיים ונהיו צ"ח חלקנום על הי"א ונהיה החלק ח' ונשארו י' אשר לא נתחלקו השיבונו אותם אל פרטים שהם י"ב בדינר ונהיו ק"כ חלקנום אל י"א ונהיה [החלק הא' י' פרטים ונשארו עוד י' בלתי מתחלקים השיבונום אל פרטי פרטים שהם גם הם י"ב בפרוטה ונהיו ק"כ חלקנום על י"א ונהיה]‫<ref>P1031 marg.</ref> החלק האחד י' ונשארו י' בלתי מתחלקים ומפני שאין בם חששא הנחנום וידענו מזה שהחלק הא' הוא ח' דינרים וי' פרוטות מי"ב בדינר וי' פרטי פרוטות מי"ב פרוטות ודבר שאין בו חששא וזהו הריוח אשר יתנו הי"ד דינרים |
| − | + | |- | |
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{X=\frac{7\sdot14}{11}=\frac{98}{11}=8+\frac{10}{11}=8+\frac{\frac{120}{11}}{12}=8+\frac{10}{12}+\frac{\frac{10}{11}}{12}=8+\frac{10}{12}+\frac{\frac{120}{11}}{12\sdot12}=8+\frac{10}{12}+\frac{10}{12\sdot12}+\frac{\frac{10}{11}}{12\sdot12}}}</math> | ||
|- | |- | ||
| − | | | + | |These are called "proportional triad" [lit. having three figures = rule of three]. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואלה הם אשר יקראו אותם בעלי שלשת הצורות |
|- | |- | ||
| − | | | + | |There are, however, other problems, which are called "proportional pentad" [lit. having five figures], in which the one who asks adds one matter to each of the ratios, such as time etc. We write them as follows: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אולם יש שאלות אחרות אשר יקראו אותם בעלי חמש צורות והם אשר ישתף השואל דבר אחד עם כל אחד מהיחסים כגון זמן וכיוצא בו ואז נכתבם ככה |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *Example: If one hires six people to work with him for three days at 8 dinar and four work with him for two days, how much would they be paid? |
| + | |style="text-align:right;"|<big>דמיון</big> אם אדם שכר ששה אנשים לעבוד עמו שלשה ימים בח' דינרים ועבדו עמו הארבעה בשני ימים כמה יקחו | ||
|- | |- | ||
| − | | | + | |Although there is no doubt that it consists of two proportions of proportional triad, the arithmeticians present a way to find all in one answer, in order to ease the one who calculates, as if it is one proportion, and they call it "proportional pentad" [lit. having five figures]. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אע"פ שאין ספק שזה מחובר משני ערכים אל שלש צורות אבל בעלי החשבון נתנו דרך למצא הכל בתשובה אחת כדי להקל על המחשב כאלו הוא ערך אחד וקראו אותו בעל חמש צורות |
|- | |- | ||
| | | | ||
| − | + | :We do as follows: | |
| − | : | + | |style="text-align:right;"|ונעשה ככה |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | |||
| − | |||
| − | |||
| | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
|- | |- | ||
| | | | ||
| − | |style=" | + | {|style="margin-left: auto; margin-right: 0px; border-collapse: collapse;" |
| − | |||
|- | |- | ||
| − | | | + | |0||2||4||8||3||6 |
| − | | | + | |} |
|- | |- | ||
| − | | | + | |} |
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :We multiply the first extreme by the days next to it and the result is the denominator by which we divide the rest, as we shall see: |
| + | |style="text-align:right;"|‫<ref>37r</ref>אנו כופלים את הקצה הראשון עם הימים אשר בצדו ומה שיהיה הוא היסוד אשר אנו חולקים עליו את השאר כאשר נראה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :We multiply the six by the three; it is 18 and this is the denominator. Then, we multiply the 8 by 4; it is 32; also the 32 by the 2; it is 64. We divide the 64 by the 18; the quotient is 3 and 10 remains undivided. We decompose the 10 into peruṭot, which are 12 in one unit; they are 120. We divide them by 18; the quotient is 6 peruṭot and 12 remain undivided. We decompose the 12 into peruṭot of peruṭot, which are 12 in one unit; they are 144. We divide them by 18; the quotient is 8. We know from this that the four people who worked two days will receive 3 dinar, 6 peruṭot, and 8 peruṭot of peruṭot, according to the condition he prescribed that the six people who will work with him for three days will receive 8 dinar. |
| + | |style="text-align:right;"|כפלנו הששה בשלשה ונהיו י"ח והוא היסוד אחר כפלנו הח' בד' ונהיו ל"ב עוד הל"ב בב' ונהיו ס"ד חלקנו [הס"ד]‫<ref>P1031 om.</ref> על הי"ח ונהיה החלק הא' ג' ונשארו גם י' בלתי מתחלקים השיבונו הי' אל הפרוטות אשר הם י"ב באחד ונהיו ק"כ חלקנום על הי"ח ונהיה החלק הא' ו' פרוטות ונשארו גם י"ב בלתי מתחלקים השיבונו הי"ב אל פרוטי פרוטות אשר הם י"ב באחד ונהיו קמ"ד חלקנום אל הי"ח ונהיה החלק הא' ח' וידענו מזה שהד' אנשים בשני ימים אשר עבדו יקחו ג' דינרים וו' פרוטות [וח' פרוטי פרוטות]‫<ref>P1031 om.</ref> לפי חשבון התנאי אשר התנה לעבוד עמו הו' אנשי' בשלשה ימים ולקחת ח' דינרים | ||
|- | |- | ||
| − | | | + | |colspan=2| |
| − | + | :<math>\scriptstyle{\color{blue}{\frac{8\sdot4\sdot2}{6\sdot3}=\frac{32\sdot2}{18}=\frac{64}{18}=3+\frac{10}{18}=3+\frac{\frac{120}{18}}{12}=3+\frac{6}{12}+\frac{\frac{12}{18}}{12}=3+\frac{6}{12}+\frac{\frac{144}{18}}{12\sdot12}=3+\frac{6}{12}+\frac{8}{12\sdot12}}}</math> | |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | |
| + | === The Separate Part: Discusses Fractions === | ||
| + | |||
| + | |style="text-align:right;"|<big>החלק הנפרד</big> המדבר על הנשברים | ||
|- | |- | ||
| − | | | + | |Said Mordecai: since the number is a discontinuous quantity and the fractions are continuous quantity, and the discontinuous and the continuous are two opposites, for one is endlessly subject to addition but not endlessly subject to division, because it stops [when reaching] one, while the other is endlessly subject to division, because the magnitude cannot be composed of parts that are indivisible. |
| − | |style="text-align:right;"|<big> | + | |style="text-align:right;"|<big>אמר מרדכי</big> להיות שהחשבון הוא מהכמה המתחלק והשברים הם שבר הכמה המתדבק והמתחלק עם המתדבק הם שני הפכים כי זה יקבל התוספת אל בלתי תכלית ולא יקבל החלוקה אל בלתי תכלית כי יעמוד באחד וזה יקבל החלוקה אל בלתי תכלית כי אי אפשר להיות הגודל מחובר מחלקים אשר לא יתחלקו |
|- | |- | ||
| − | | | + | |Therefore, the rules of fractions are the opposite of the rules of integers. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|לכן היה משפטי השברים הפך משפטי השלמים |
|- | |- | ||
| − | | | + | |For, the product of integers is greater than the multiplicands, while the product of fractions is smaller than the multiplicands. |
| − | |style="text-align:right;"|< | + | |style="text-align:right;"|כי כפל השלמים הוא יותר מהמספרים אשר יכפלו [וכפל השברי' הוא פחות מהממספרי' אשר יכפלו]‫<ref>P1031 om.</ref> |
|- | |- | ||
| − | | | + | |Since one is mean between the integers and the fractions, its product is equal to itself, because it is drawn to both sides equally. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ומפני שהאחד הוא אמצעי בין השלמים ובין השברים לכן היה כפלו כמוהו כי ימשך אל שתי צדדיו בשווי |
|- | |- | ||
| − | | | + | |Since the magnitude of the fractions agrees with the parts that are summed in a whole unit, they borrowed their name, for the half is derived from two, the third from 3, the quarter from 4, so the denominator is derived from them, as we will explain. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ומפני שהשברים בגודל יסכימו עם החלקים שהם בכלל אחד מקובץ לקחו השם מהם כי החצי נגזר משנים והשלישית מג' והרביעית מד' ולכן יהיה המורה מהם כאשר נבאר |
|- | |- | ||
| − | + | |They are divided by the square of the denominator and the required is found. | |
| − | + | |style="text-align:right;"|אחר יחלקו על מרובע [המורה] ‫<ref>P1031: המדה</ref> וימצא המבוקש | |
|- | |- | ||
| + | !<span style=color:Green>Multiplication of Fractions</span> | ||
| | | | ||
| − | |||
|- | |- | ||
| | | | ||
| − | *Example: | + | *Example: one asks: how much is the product of a third by a third? |
| − | :<math>\scriptstyle\frac{ | + | :<math>\scriptstyle\frac{1}{3}\times\frac{1}{3}</math> |
| − | |style="text-align:right;"|<big>דמיון</big> שאל | + | |style="text-align:right;"|<big>דמיון</big> שאל השואל כפל שלישית על שלישית כמה יהיה |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::The denominator is 9, because we multiply 3 by 3. |
| + | ::<math>\scriptstyle{\color{blue}{3\sdot3=9}}</math> | ||
| + | |style="text-align:right;"|והנה המורה ט' כי כפלנו ג' על ג‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Its square is 81. |
| + | ::<math>\scriptstyle{\color{blue}{9^2=81}}</math> | ||
| + | |style="text-align:right;"|ומרבעו פ"א | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :We divide the denominator by its square; the result is one ninth and from this we know that the product of a third by a third is a ninth. |
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{3}\times\frac{1}{3}=\frac{9}{81}=\frac{1}{9}}}</math> | ||
| + | |style="text-align:right;"|חלקנו המורה על מרובעו ועלה תשיעי' אחת ומזה ידענו שכפל שלישית על שלישית יהיה תשיעית | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *Also if they are two thirds by two thirds |
| + | :<math>\scriptstyle\frac{2}{3}\times\frac{2}{3}</math> | ||
| + | |style="text-align:right;"|וכן אם היו ב' שלישיות על ב' שלישיות | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::The denominator is 9, because we multiply three by three, so it is 9. |
| + | ::<math>\scriptstyle{\color{blue}{3\sdot3=9}}</math> | ||
| + | |style="text-align:right;"|המורה ט' כי כפלנו שלש על שלש הם ט‫' | ||
|- | |- | ||
| | | | ||
| − | + | ::Its square is 81. | |
| − | :<math>\scriptstyle | + | ::<math>\scriptstyle{\color{blue}{9^2=81}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ומרובעו פ"א |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Since the thirds are 2 by 2, we take 6 for the 2-thirds and 6 for the other 2-thirds. We multiply 6 by 6; it is 36. |
| + | ::<math>\scriptstyle{\color{blue}{6\sdot6=36}}</math> | ||
| + | |style="text-align:right;"|ומפני שהשלישיות היו ב' על ב' לקחנו בעד הב' שלישיות [ו' וכן ו' בעד הב' שלישיות]‫<ref>P1031 om.</ref> האחרים כפלנו ו' על ו' ונהיו ל"ו | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :We divide it by 81; the quotient is a third and a ninth and this is the required. |
| + | :<math>\scriptstyle{\color{blue}{\frac{2}{3}\times\frac{2}{3}=\frac{36}{81}=\frac{1}{3}+\frac{1}{9}}}</math> | ||
| + | |style="text-align:right;"|חלקנום על פ"א ונהיה החלק האחד שלישית ותשיעית וזהו המבוקש | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *Another example: one asks: how much are 3 quarters multiplied by 4 ninths? |
| + | :<math>\scriptstyle\frac{3}{4}\times\frac{4}{9}</math> | ||
| + | |style="text-align:right;"|<big>דמיון</big> אחר השואל כמה ג' רביעיות הנכפלות על ארבע תשיעיות | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::The denominator is 36, because the product of four by 9 is 36. |
| + | ::<math>\scriptstyle{\color{blue}{4\sdot9=36}}</math> | ||
| + | |style="text-align:right;"|הנה המורה ל"ו כי כפל הארבעה על הט' הם ל"ו | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Its square is 1296. |
| + | ::<math>\scriptstyle{\color{blue}{36^2=1296}}</math> | ||
| + | |style="text-align:right;"|ומרבעו אלף רצ"ו | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Since the quarters are 3, we take 12 for them, because 3 times 4 is 12. Since the ninths are 4, we take 36 for them. We multiply 12 by 36; it is 432. |
| + | ::<math>\scriptstyle{\color{blue}{12\sdot36=432}}</math> | ||
| + | |style="text-align:right;"|ומפני כי הרביעיות היו ג' לקחנו י"ב בעדם כי ג' פעמים ד' הם י"ב ומפני שהתשיעיות הם ד' לקחנו בעדם ל"ו הכינו הי"ב עם הל"ו ונהיו תל"ב | ||
|- | |- | ||
| − | |||
| | | | ||
| + | :We divide it by the square of the denominator, which is 1296; the quotient is one third and from this we know that the required is one third. | ||
| + | :<math>\scriptstyle{\color{blue}{\frac{3}{4}\times\frac{4}{9}=\frac{432}{1296}=\frac{1}{3}}}</math> | ||
| + | |style="text-align:right;"|חלקנום על מרובע המורה שהם אלף רצ"ו ונהיה החלק האחד שלישית אחד ומזה ידענו שהמבוקש הוא שלישית אחד | ||
|- | |- | ||
| − | | | + | |However, the arithmeticians extract the denominator in an easier way, because this denominator is a large number, so they do like that: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אבל בעלי החשבון לוקחים המורה באופן יותר נקל כי זה המורה הוא רב החשבון ועושים ככה |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :When they are asked about the multiplication of a third by a third, they write the one above, then they draw a line and write the three below. They do the same with the other third. According to this diagram. |
| + | |style="text-align:right;"|כשישאלו על כפל שלישית אחד על שלישית אחד כותבים האחד למעלה ואחר הם מתווכים עם קו וכותבים השלישית למטה וככה יעשו בשלישית האחרת כזאת הצורה | ||
|- | |- | ||
| | | | ||
| − | |||
| − | |||
| | | | ||
| − | |style="text-align: | + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" |
|- | |- | ||
| | | | ||
| − | |style=" | + | {|style="margin-left: auto; margin-right: 0px; border-collapse: collapse;" |
|- | |- | ||
| − | | | + | |<math>\scriptstyle\frac{1}{3}</math>||<math>\scriptstyle\frac{1}{3}</math> |
| − | | | + | |} |
|- | |- | ||
| − | | | + | |} |
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :They multiply the one by the one; it is one. They multiply the 3 by the 3; it is 9. Then, they examine what is the ratio of 1 to 9 and it is the required. |
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{3}\times\frac{1}{3}=\frac{1\sdot1}{3\sdot3}=1:9}}</math> | ||
| + | |style="text-align:right;"|וכופלים האחד על האחד ונהיה אחד אחר כן יכפלו הג' על הג' ונהיו ט' אחר רואים מה ערך הא' אל הט' והוא המבוקש | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *Another example: how much are 2 thirds multiplied by 4 quarters? |
| + | :<math>\scriptstyle\frac{2}{3}\times\frac{4}{4}</math> | ||
| + | |style="text-align:right;"|<big>דמיון</big> אחר כמה ב' שלישיות הכפולות על ד' רביעיות | ||
|- | |- | ||
| − | |||
| | | | ||
| + | :We write the 2 above and the three below and draw a line between them. We do the same with the 4-quarters. Like this: | ||
| + | |style="text-align:right;"|כתבנו הב' למעלה ‫<ref>37v</ref>והשלישיות למטה והתווכנו עם קו אחד וכן עשינו בד' הרביעיות כזה | ||
|- | |- | ||
| | | | ||
| − | |||
| − | |||
| | | | ||
| − | |style="text-align: | + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" |
|- | |- | ||
| | | | ||
| − | |style=" | + | {|style="margin-left: auto; margin-right: 0px; border-collapse: collapse;" |
| + | |- | ||
| + | |<math>\scriptstyle\frac{4}{4}</math>||<math>\scriptstyle\frac{2}{3}</math> | ||
| + | |} | ||
|- | |- | ||
| − | |||
| − | |||
|} | |} | ||
| − | |||
|- | |- | ||
| | | | ||
| − | + | :We multiply 2 by 4; it is 8. We multiply also the 3 below by the 4 below; it is 12. Then, we examine what is the ratio of 8 to 12; it is two-thirds. From this we know that the required is two-thirds of one. | |
| − | + | :<math>\scriptstyle{\color{blue}{\frac{2}{3}\times\frac{4}{4}=\frac{2\sdot4}{3\sdot4}=8:12=\frac{2}{3}}}</math> | |
| − | + | |style="text-align:right;"|כפלנו [הב' עם הד' ונהיו ח' עוד כפלנו]‫<ref>P1031 om.</ref> הג' שלמטה עם הד' שלמטה ונהיו י"ב אחר עייננו מה ערך הח' אל הי"ב והם שני שלישים וידענו מזה שהמבוקש הם שני שלישיות אחד | |
| − | |||
|- | |- | ||
| − | + | |If we want to multiply integers and fractions by integers and fractions: | |
| − | | | + | |style="text-align:right;"|ואם רצינו לכפול שלמים ונשברים עם שלמים ונשברים |
|- | |- | ||
| − | | | + | |We write them like this: the integers and next to the them fractions; the same for those by which we want to multiply them. |
| − | : | + | |style="text-align:right;"|אנו כותבים אותם ככה השלמים ובצדם הנשברים וכן אשר נרצה לכפול עליהם |
| − | |||
| − | |style="text-align:right;"| | ||
|- | |- | ||
| − | | | + | |Then, we decompose the integers to fractions as follows: we multiply the parts of the continuous by the integers as if they are also integers and add the number of the parts to them also, so they are all become fractions, like this: |
| − | + | |style="text-align:right;"|אחר אנו משברים השלמים בכזה האופן אנו מכים חלקי המתדבק עם השלמים כמו שאם היו גם הם שלמים ואנו מחברים גם מספר החלקים עמהם ואז שבו כלם נשברים כזה | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | *How much are 5 integers and 3-quarters multiplied by 4 integers and 2-thirds? | |
| − | + | :<math>\scriptstyle\left(5+\frac{3}{4}\right)\times\left(4+\frac{2}{3}\right)</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כמה הם חמשה שלמים ושלשת רביעיות הכפולים על ד' שלמים וב' שלישיות |
|- | |- | ||
| | | | ||
| − | : | + | :This is the diagram: |
| − | + | |style="text-align:right;"|והנה הצורה | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | |||
| − | |||
| − | |||
| − | |||
| | | | ||
| − | + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | |
| − | |style="text-align: | ||
|- | |- | ||
| | | | ||
| − | + | {|style="margin-left: auto; margin-right: 0px; border-collapse: collapse;" | |
| − | |||
| − | |style=" | ||
|- | |- | ||
| − | | | + | |<math>\scriptstyle\frac{3}{4}</math>||5 |
| − | |||
| − | |||
| − | | | ||
|- | |- | ||
| − | | | + | |<math>\scriptstyle\frac{2}{3}</math>||4 |
| − | + | |} | |
| − | |||
| − | | | ||
|- | |- | ||
| − | | | + | |} |
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | :: | + | ::We multiply the four, which is the denominator of the continuous part, by the five integers; they become 20. We add also the 3, which is the number of the quarters; it is 23-quarters. |
| − | ::<math>\scriptstyle{\color{blue}{\frac{ | + | ::<math>\scriptstyle{\color{blue}{5+\frac{3}{4}=\frac{\left(4\sdot5\right)+3}{4}=\frac{20+3}{4}=\frac{23}{4}}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|הכינו הארבעה שהם מורה החלק המתדבק עם החמשה שלמים ושבו כ' חברנו גם הג' שהם מספר הרביעיות ונהיו כ' וג' רביעיות |
|- | |- | ||
| − | |||
| | | | ||
| + | ::We multiply the 3, which is the denominator of the continuous part, by the 4 integers; they become 12. We add to it also the two; it is 14-thirds. | ||
| + | ::<math>\scriptstyle{\color{blue}{4+\frac{2}{3}=\frac{\left(3\sdot4\right)+2}{3}=\frac{12+2}{3}=\frac{14}{3}}}</math> | ||
| + | |style="text-align:right;"|עוד הכינו הג' שהם מורה חלקי המתדבק עם הד' השלמי' ונהיו י"ב חברנו גם השנים עמהם ונהיו י"ד שלישיות | ||
|- | |- | ||
| − | |||
| | | | ||
| + | ::So, the former are 23-quarters and these are 14-thirds. We multiply these by those; it is 322. | ||
| + | ::<math>\scriptstyle{\color{blue}{23\sdot14=322}}</math> | ||
| + | |style="text-align:right;"|והנה הראשונים כ"ג רביעיות ואלה י"ד שלישיות הכינו אלה עם אלה והיו שכ"ב | ||
|- | |- | ||
| | | | ||
| − | : | + | ::We multiply also the 4 by the 3, which are the denominators of the thirds and the quarters; it is 12. |
| − | : | + | ::<math>\scriptstyle{\color{blue}{4\sdot3=12}}</math> |
| − | :<math>\scriptstyle | + | |style="text-align:right;"|עוד הכינו הד' עם הג' שהם מורי השלישיות והרביעיות ונהיו י"ב |
| − | |style="text-align:right;"| | ||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :We examine what is the ratio of 322 to 12; it is 27 minus a sixth. From this we know that the product of 5 integers and 3-quarters by 4 integers and 2-thirds is 27 minus a sixth. |
| − | + | :<math>\scriptstyle{\color{blue}{\left(5+\frac{3}{4}\right)\times\left(4+\frac{2}{3}\right)=322:12=27-\frac{1}{6}}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ראינו מה ערך השכ"ב אל הי"ב והם כ"ז פחות ששית וידענו מזה כי כפל ה' שלמים וג' רביעיות על ד' שלמים וב' שלישיות הם כ"ז פחות ששית |
|- | |- | ||
| − | | | + | |This is the method the arithmeticians use when there are fractions with integers, whether they are integers alone by integers and fractions, or integers and fractions by integers and fractions, or integers alone by fractions alone. |
| − | :: | + | |style="text-align:right;"|זאת היא הדרך אשר נהגו בעלי החשבון להתנהג בהיות שם שברים עם שלמים בין שהיו שלמים לבד עם שלמים ושברים ובין שהיו שלמים ושברים [עם שלימי' ושברי']‫<ref>P1031 om.</ref> ובין שהיו שלמים לבד עם שברים לבד |
| − | + | |- | |
| − | |style="text-align:right;"| | + | |When there are integers alone by fractions alone, there is also another way: that we take for the integers a denominator as their number, we multiply it by the numerator of the fraction, then divide it by the denominator of the fraction; the result is the required. |
| + | |style="text-align:right;"|אמנם בהיות שלמים לבד עם שברים לבד יש גם כן דרך אחרת והיא שנקח בעבור השלמי' מורה כמספרם ונכה אותו עם מספר חלקי השברים ונחלקהו על המורה שנקח מהשברי' והעולה הוא המבוקש | ||
|- | |- | ||
| | | | ||
| − | ::< | + | *Example: how much are 2 integers multiplied by 3-quarters? |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle2\times\frac{3}{4}</math> |
| + | |style="text-align:right;"|<big>דמיון</big> כמה הם ב' שלמים הכפולים על ג' רביעיות | ||
|- | |- | ||
| | | | ||
| − | : | + | :We take 2 for the 2 integers and this is their denominator. We multiply it by 3, which is the numerator of the fraction; it is 6. We divide it by four, which is the denominator of the fraction; it is one and a half and this is the required. |
| − | + | :<math>\scriptstyle{\color{blue}{2\times\frac{3}{4}=\frac{2\sdot3}{4}=\frac{6}{4}=1+\frac{1}{2}}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|לקחנו בעד הב' שלמים ב' והוא המורה שלהם כפלנום עם ג' שהוא מספר חלקי השברים נהיו ו' חלקנום על ארבעה שהוא המורה שלקחנו מהשברים ונהיו אחד וחצי וזהו המבוקש |
|- | |- | ||
| − | !<span style=color:Green> | + | !<span style=color:Green>Proportions of Fractions</span> |
| | | | ||
| + | |- | ||
| + | |If you want to relate fractions to fractions, whether there are integers with each of them, or with one of them, you should decompose the integers to fractions first and convert them to fractions according to the type of the fractions that are summed with them. | ||
| + | |style="text-align:right;"|ואם רצית לערוך שברים על שברים בין שיהיו שלמים עם כל אחד מהם [או עם האחד]‫<ref>P1031 om.</ref> לבד ראוי בתחלה לשבור השלמים ולעשותם שברים כפי המין אשר היו השברים הדבקים עמהם | ||
|- | |- | ||
| | | | ||
| − | : | + | :Write them as follows: |
| − | + | |style="text-align:right;"|וככה תכתבם | |
| − | |style="text-align:right;"| | ||
| − | |||
|- | |- | ||
| | | | ||
| − | + | | | |
| − | |style="text-align: | + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" |
|- | |- | ||
| | | | ||
| − | :: | + | {|style="margin-left: auto; margin-right: 0px; border-collapse: collapse;" |
| − | + | |- | |
| − | | | + | |0||<math>\scriptstyle\frac{3}{6}</math>||4||<math>\scriptstyle\frac{4}{5}</math>||3||<math>\scriptstyle\frac{2}{3}</math>||2 |
| + | |} | ||
| + | |- | ||
| + | |} | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::First, multiply the two integers by the three, which is the denominator of the thirds; it is 6. Then, add to it also the 2, which is the number of the thirds; it is 8-thirds. |
| − | ::<math>\scriptstyle{\color{blue}{ | + | ::<math>\scriptstyle{\color{blue}{2+\frac{2}{3}=\frac{\left(2\sdot3\right)+2}{3}=\frac{6+2}{3}=\frac{8}{3}}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|תכה בתחלה השני שלמים עם השלש שהוא מורה השלישיות ונהיו ו' אחר תחבר גם הב' עמהם שהוא מספר השלישיות ונהיו ח' שלישיות |
|- | |- | ||
| | | | ||
| − | :: | + | ::We multiply the 3 integers by the 5, which is the denominator of the fifths; it is 15. We add to it also the 4, which is the number of the fifths; it is nineteen fifths. |
| − | ::<math>\scriptstyle{\color{blue}{ | + | ::<math>\scriptstyle{\color{blue}{3+\frac{4}{5}=\frac{\left(3\sdot5\right)+4}{5}=\frac{15+4}{5}=\frac{19}{5}}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|עוד הכינו הג' שלמים עם הה' שהוא מורה החמישיות ונהיו ט"ו חברנו גם הד' עמהם שהם מספר החמישיות ונהיו תשע עשרה חמישיות |
|- | |- | ||
| | | | ||
| − | ::We | + | ::We multiply the 4 integers by the 6, which is the denominator of the sixths; it is 24. We add to it also the 3, which is the number of the sixths; it is 27-sixths. |
| − | ::<math>\scriptstyle{\color{blue}{ | + | ::<math>\scriptstyle{\color{blue}{4+\frac{3}{6}=\frac{\left(4\sdot6\right)+3}{6}=\frac{24+3}{6}=\frac{27}{6}}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|עוד הכינו הד' שלמים עם הו' שהם מורה הששיות ונהיו כ"ד חברנו גם הג' שהם מספר הששיות עמהם ונהיו כ"ז ששיות |
|- | |- | ||
| | | | ||
| − | + | *For, the problem is: if 2 measures and 2-thirds give us 3 dinar and 4-fifths, how much will 4 measures and three-sixths give [us]? | |
| − | : | + | :<math>\scriptstyle\left(2+\frac{2}{3}\right):\left(3+\frac{4}{5}\right)=\left(4\frac{3}{6}\right):X</math> |
| − | :<math>\scriptstyle | + | |style="text-align:right;"|כי השאלה היתה אם הב' מדות וב' שלישיות יתנו לנו ג' דינרים וד' חמישיות הד' מדות ושלש ששיות כמה יתנו |
| − | |style="text-align:right;"| | ||
| − | כמה | ||
|- | |- | ||
| | | | ||
| − | :: | + | :It becomes now: if 8-thirds give us 19-fifths, how much will 27-sixths give [us]? |
| − | + | :<math>\scriptstyle\frac{8}{3}:\frac{19}{5}=\frac{27}{6}:X</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ושבה השאלה עתה אם הח' שלישיות יתנו לנו י"ט חמשיות הכ"ז ששיות כמה יתנו |
|- | |- | ||
| | | | ||
| − | : | + | :After we convert the problem into fractions, we write them as follows: |
| − | : | + | |style="text-align:right;"|ואחר שהשיבונו השאלה אל השברים אנו כותבים אותם כך |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | | | |
| − | |style="text-align: | + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" |
|- | |- | ||
| | | | ||
| − | :: | + | {|style="margin-left: auto; margin-right: 0px; border-collapse: collapse;" |
| − | |style=" | + | |-style="font-size: 75%;" |
| + | |​||​||​||57||​ | ||
| + | |- | ||
| + | |<math>\scriptstyle\frac{1539}{240}</math>||0||<math>\scriptstyle\frac{27}{6}</math>||<math>\scriptstyle\frac{19}{5}</math>||<math>\scriptstyle\frac{8}{3}</math> | ||
| + | |-style="font-size: 75%;" | ||
| + | |​||​||​||40||​ | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::Then, we multiply them in the form of scissors: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אחר כן אנו מכים אותם כדמות מספריים |
|- | |- | ||
| | | | ||
| − | ::We | + | ::We multiply 8 by 5; it is 40. We write it beneath the 5. |
| − | ::<math>\scriptstyle{\color{blue}{ | + | ::<math>\scriptstyle{\color{blue}{8\sdot5=40}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואנו מכים הח' עם הה' ונהיו מ' ואנו כותבים אותם ‫<ref>38r</ref>תחת הה‫' |
|- | |- | ||
| − | |||
| | | | ||
| + | ::We multiply 40 by the 6; it is 240. We write it beneath the 6 and this is the denominator by which we divide. | ||
| + | ::<math>\scriptstyle{\color{blue}{40\sdot6=240}}</math> | ||
| + | |style="text-align:right;"|עוד אנו מכים המ' עם הו' ונהיו ר"מ ואנו כותבים תחת הו' וזהו המספר אשר נחלוק עליו | ||
|- | |- | ||
| | | | ||
| − | : | + | ::We start multiplying 3 by 19; it is 57. We write it above the 19. |
| − | + | ::<math>\scriptstyle{\color{blue}{3\sdot19=57}}</math> | |
| − | : | + | |style="text-align:right;"|עוד אנו מתחילים להכות הג' עם הי"ט ונהיו נ"ז ואנו כותבים אותם על הי"ט |
| − | :<math>\scriptstyle | + | |- |
| − | |style="text-align:right;"| | + | | |
| − | + | ::We multiply 57 by 27; it is 1539. | |
| − | + | ::<math>\scriptstyle{\color{blue}{57\sdot27=1539}}</math> | |
| + | |style="text-align:right;"|עוד אנו מכים הנ"ז עם הכ"ז ונהיו אלף תקל"ט | ||
|- | |- | ||
| | | | ||
| − | : | + | :Then, we divide it by 240; it is 6 integers and 2-fifths and one part of eighty and this is the required that gives us the wanted amount. |
| − | + | :<math>\scriptstyle{\color{blue}{X=\frac{1539}{240}=6+\frac{2}{5}+\frac{1}{80}}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אחר כן אנו מחלקים אותם על הר"מ ונהיו ו' שלמי' וב' חמישיות וחלק אחד משמנים וזהו המבוקש אשר יתן לנו החשבון הדרוש |
| + | |- | ||
| + | |Yet, if the problem involves proportional pentad, for instance, when the one who asks adds another thing to his question, like time etc., as we have explained for integers, then we write as follows: | ||
| + | |style="text-align:right;"|אבל אם החשבון יהיה בעל חמש צורות כגון שישתף השואל דבר אחר עם שאלתו כגון זמן וכדומה לו כאשר בארנו בשלמים אז נכתוב ככה | ||
|- | |- | ||
| | | | ||
| − | : | + | *Example: one asks if 2-quarters of a dinar in 4-eighths of a day, which are 12 hours, because an eighth of a day is 3 hours, earn 3-quarters of a dinar, how much will 4-quarters earn in 4-eighths? |
| − | + | |style="text-align:right;"|<big>דמיון</big> שאל השואל אם ב' רביעיות דינר בד' שמיניות היום שהם י"ב שעות כי שמינית היום ג' שעות הרויחו ג' רביעיות דינר הד' רביעיות בד' שמיניות היום כמה ירויחו | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | :: | + | :This is its diagram: |
| − | + | |style="text-align:right;"|וזה צורתו | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | | | |
| − | + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | |
| − | |||
|- | |- | ||
| − | |||
| | | | ||
| + | {|style="margin-left: auto; margin-right: 0px; border-collapse: collapse;" | ||
| + | |-style="font-size: 75%;" | ||
| + | |​||<span style=color:red>1536</span>||384||96||colspan=2|8 | ||
| + | |- | ||
| + | |0||<math>\scriptstyle\frac{4}{8}</math>||<math>\scriptstyle\frac{4}{4}</math>||<math>\scriptstyle\frac{3}{4}</math>||<math>\scriptstyle\frac{4}{8}</math>||<math>\scriptstyle\frac{2}{4}</math> | ||
| + | |-style="font-size: 75%; text-align:right;" | ||
| + | |​||1024||32||​||colspan=2|32 | ||
| + | |-style="font-size: 75%; text-align:right;" | ||
| + | |​||​||​||​||colspan=2|4 | ||
| + | |-style="font-size: 75%; text-align:right;" | ||
| + | |​||​||​||​||colspan=2|128 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
|- | |- | ||
| | | | ||
| − | : | + | ::We multiply 2 by the 4 in the upper line, which are the numbers of the quarters and the eighths; it is 8. |
| − | : | + | ::<math>\scriptstyle{\color{blue}{2\sdot4=8}}</math> |
| − | :<math>\scriptstyle | + | |style="text-align:right;"|אז נכפול הב' עם הד' שבטור העליון והם מספר הרביעיות והשמיניות ונהיו ח‫' |
| − | |style="text-align:right;"| | ||
| − | |||
|- | |- | ||
| | | | ||
| − | :: | + | ::Then, we multiply 8 by the 4 in the bottom line; it is 32. We write it beneath it. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{8\sdot4=32}}</math> |
| + | |style="text-align:right;"|אחר הכינו הח' עם הד' שבטור השפל ונהיו ל"ב וכתבנום תחתיהם | ||
|- | |- | ||
| | | | ||
| − | ::We | + | ::We multiply 32 by the 4 next to it, beneath the 4 in the upper line; it is 128. We write it beneath it. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{32\sdot4=128}}</math> |
| + | |style="text-align:right;"|עוד הכינו הל"ב עם הד' אשר בצדם שהם תחת הד' שבטור העליון ונהיו קכ"ח וכתבנום תחתיהם | ||
|- | |- | ||
| | | | ||
| − | ::We multiply the | + | ::We multiply 128 by the eight next to it; it is 1024. We write it beneath it and this is the denominator by which we divide. |
| − | |style="text-align:right;"|עוד | + | ::<math>\scriptstyle{\color{blue}{128\sdot8=1024}}</math> |
| + | |style="text-align:right;"|עוד הכינו הקכ"ח עם השמנה אשר בצדם ונהיו אלף כ"ד וכתבנום תחתיהם ואלה הם היסוד אשר נחלוק עליהם | ||
|- | |- | ||
| | | | ||
| − | ::We | + | ::We multiply 4 by the 8 in the bottom line; it is 32. We write it beneath them. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{8\sdot4=32}}</math> |
| + | |style="text-align:right;"|עוד אנו מכים הד' עם הח' שבטור השפל ונהיו ל"ב ואנו כותבי' אותם תחתיהם | ||
|- | |- | ||
| | | | ||
| − | ::We | + | ::We multiply 32 by the 3 in the upper line; it is 96. We write it above it. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{32\sdot3=96}}</math> |
| + | |style="text-align:right;"|עוד אנו מכים הל"ב עם הג' שבטור העליון ונהיו צ"ו ואנו כותבים אותם עליהם | ||
|- | |- | ||
| | | | ||
| − | ::We | + | ::We multiply 96 by the 4 next to it; it is 384. We write it above it. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{96\sdot4=384}}</math> |
| + | |style="text-align:right;"|עוד אנו מכים הצ"ו עם הד' אשר בצדם ונהיו שפ"ד ואנו כותבים אותם עליהם | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\ | + | ::We multiply 386 by the 4 next to it; it is 1536. We write it above it. |
| + | ::<math>\scriptstyle{\color{blue}{384\sdot4=1536}}</math> | ||
| + | |style="text-align:right;"|עוד אנו מכים השפ"ד עם הד' אשר בצדם ונהיו אלף תקל"ו ואנו כותבים אותם עליהם | ||
| + | |- | ||
| | | | ||
| + | :Then, we divide it by 1024; the resulting quotient is one and a half. From this we know that the wanted amount that the 4-quarters earn in 4-eighths of a day is 1 and a half dinar. | ||
| + | :<math>\scriptstyle{\color{blue}{X=\frac{1536}{1024}=1+\frac{1}{2}}}</math> | ||
| + | |style="text-align:right;"|אחר כן אנו מחלקים אותם על האלף וכ"ד ויצא החלק אחד וחצי וידענו מזה שזהו החשבון המבוקש אשר ירויחו הד' רביעיות בד' שמיניות היום כי ירויחו א' וחצי דינר | ||
|- | |- | ||
| | | | ||
| − | : | + | *Another example: we wish to know if 2-thirds of a dinar in 4-fifths of a day earn 6-sevenths, how much will 8-ninths earn in 10-tenths? |
| − | + | |style="text-align:right;"|<big>דמיון</big> אחר לזה רצינו לדעת אם הב' שלישיות דינר בד' חמישיות היום ירויחו ו' שביעיות הח' תשיעיות בי' עשיריות כמה יתנו | |
| − | |||
| − | |style="text-align:right;"|<big> | ||
| − | כמה | ||
|- | |- | ||
| | | | ||
| − | :: | + | :We write them like this: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכתבנום ככה |
|- | |- | ||
| | | | ||
| − | + | | | |
| − | + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | |
| − | |||
|- | |- | ||
| | | | ||
| − | :: | + | {|style="margin-left: auto; margin-right: 0px; border-collapse: collapse;" |
| − | ::<math>\scriptstyle{\ | + | |-style="font-size: 75%;" |
| − | |style=" | + | |​||<span style=color:red>7200</span>||<span style=color:red>720</span>||<span style=color:red>90</span>||colspan=2|8 |
| + | |- | ||
| + | |0||<math>\scriptstyle\frac{7}{10}</math>||<math>\scriptstyle\frac{8}{9}</math>||<math>\scriptstyle\frac{6}{7}</math>||<math>\scriptstyle\frac{4}{5}</math>||<math>\scriptstyle\frac{2}{3}</math> | ||
| + | |-style="font-size: 75%;" | ||
| + | |​||5040||504||56||15||​ | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
|- | |- | ||
| | | | ||
| − | : | + | ::We multiply 2 by the 4 in the upper line; it is 8. We write it above them. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{2\sdot4=8}}</math> |
| + | |style="text-align:right;"|כפלנו הב' עם הד' שבטור העליון ונהיו ח' וכתבנום עליהם | ||
|- | |- | ||
| | | | ||
| − | : | + | ::We multiply 8 by the 7 that is third in the bottom line; it is 56. We write it beneath the 7 in the bottom line. |
| − | : | + | ::<math>\scriptstyle{\color{blue}{8\sdot7=56}}</math> |
| − | :<math>\scriptstyle | + | |style="text-align:right;"|עוד הכינו הח' עם הז' שהוא שלישי לטור השפל ונהיו נ"ו וכתבנום תחת הז' שבטור השפל |
| − | |style="text-align:right;"| | ||
| − | |||
|- | |- | ||
| | | | ||
| − | :: | + | ::We multiply 56 by 9; it is 504. We write it beneath the 9. |
| − | ::<math>\scriptstyle{\color{blue}{ | + | ::<math>\scriptstyle{\color{blue}{56\sdot9=504}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|עוד הכינו הנ"ו עם הט' ונהיו תק"ד וכתבנום תחת הט‫' |
|- | |- | ||
| | | | ||
| − | :: | + | ::We multiply 504 by the 10; it is 5 thousand and 40. We write it beneath the ten and this is the denominator by which we divide. |
| − | ::<math>\scriptstyle{\color{blue}{ | + | ::<math>\scriptstyle{\color{blue}{8\sdot7=56}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|עוד הכינו התק"ד עם הי' ונהיו ה' אלפים ומ' וכתבנום תחת העשרה ואלה הם היסוד אשר נחלק עליהם |
|- | |- | ||
| | | | ||
| − | :: | + | ::We multiply 3 by the 5 in the bottom line; it is 15. We write it beneath the 5. |
| − | ::<math>\scriptstyle{\color{blue}{ | + | ::<math>\scriptstyle{\color{blue}{3\sdot5=15}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|עוד חזרנו והכינו את הג' עם הה' שבטור השפל ונהיו ט"ו וכתבנום למטה תחת הה‫' |
|- | |- | ||
| | | | ||
| − | : | + | ::We multiply 15 by the 6 that is third in the upper line; it is 90. We write it above the 6. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{15\sdot6=90}}</math> |
| + | |style="text-align:right;"|אחר הכינו את הט"ו עם הוו' שבטור העליון שהוא השלישי והיו צ' וכתבנום על הו‫' | ||
|- | |- | ||
| − | |||
| | | | ||
| + | ::We multiply 90 by the 8; it is seven hundred and 20. We write it above the 8. | ||
| + | ::<math>\scriptstyle{\color{blue}{90\sdot8=720}}</math> | ||
| + | |style="text-align:right;"|עוד הכינו הצ' עם הח' ונהיו שבע מאות וכ' וכתבנום על הח‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | ::We multiply the seven hundred and 20 by 10; it is 7 thousand and two hundred. |
| − | : | + | ::<math>\scriptstyle{\color{blue}{720\sdot10=7200}}</math> |
| − | :<math>\scriptstyle | + | |style="text-align:right;"|עוד הכינו את השבע מאות וכ' עם הי' ונהיו ז' אלפים ומאתים |
| − | |style="text-align:right;"| | ||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :We divide it by the denominator, which is 5040; the resulting quotient is one plus a third and one part of 10. From this we know that this is what the 8-ninths of a dinar earn in 10-tenths of a day, if 2-thirds of a dinar earn 6-sevenths in 4-fifths of a day. |
| − | + | :<math>\scriptstyle{\color{blue}{X=\frac{7200}{5040}{\color{red}{\approx}}1+\frac{1}{3}+\frac{1}{10}}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|חלקנו אותם על היסוד שהם ה' אלפי' מ' ונהיה החלק אחד ושליש וחלק א' מי' וידענו מזה שזהו מה שירויחו הח' תשיעיות דינר בי' עשיריות היום אם הב' שלישיות דינר הרויחו ו' שביעיות בד' חמישיות היום |
| + | |- | ||
| + | |This is the way all traders and arithmeticians use for these two types of fractions, i.e. the proportional triad and the proportional pentad. | ||
| + | |style="text-align:right;"|זהו הדרך אשר ישתמשו בה כל הסוחרים ובעלי החשבון באלה שתי מיני הצורות לשברים ר"ל בבעלי השלש צורות ובעלי החמש צורות | ||
| + | |- | ||
| + | |However there is another way, but it requires dividing three times, and it is as follows: | ||
| + | |style="text-align:right;"|אולם יש דרך אחרת גם היא נכונה אבל יצטרך לחלק שלש פעמים והיא זאת | ||
|- | |- | ||
| | | | ||
| − | : | + | *Example of the first proportional triad, the problem is: if 8-thirds yield 19-fifths, how much will 27-sixths yield? |
| − | + | :<math>\scriptstyle\frac{8}{3}:\frac{19}{5}=\frac{27}{6}:X</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<big>דמיון</big> בבעלת ‫<ref>38v</ref>השלש צורות הראשונה שהשאלה היא אם הח' שלישיות יתנו לנו י"ט חמישיות הכ"ז ששיות כמה יתנו |
| + | |- | ||
| + | |We write them according to the first rule with a separating line. We multiply the second numerator in the upper line by the third and we divide [the product] by the first. We do the same with the bottom line. Then we divide the quotient of the upper line by the quotient of the bottom line; the result is the required. | ||
| + | |style="text-align:right;"|אנו כותבים אותם כמשפט הראשון עם הקו המתויך כך אחר אנו מכים מספר החלקים שבטור העליון השני עם השלישי ואנו מחלקי' אותם על הראשון וכן נעשה גם בטור השפל אחר כן אנו מחלקים מה שיצא מן החלק בטור העליון על מה שיצא מן החלק בטור השפל והיוצא הוא המבוקש | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::Example: We multiply the 19 by the 27; it is 513. We divide it by the 8; the quotient is 64 and an eighth. We keep them. |
| − | ::<math>\scriptstyle{\color{blue}{ | + | ::<math>\scriptstyle{\color{blue}{\frac{19\sdot27}{8}=\frac{513}{8}=64+\frac{1}{8}}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<big>דמיון</big> הכינו הי"ט עם הכ"ז ונהיו תקי"ג חלקנו אותם על הח' ונהיה החלק הא' ס"ד ושמינית ושמרנום |
|- | |- | ||
| | | | ||
| − | ::We | + | ::We multiply also the 5 by the 6 in the bottom line; it is 30. We divide it by the 3; the quotient is 10. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\frac{5\sdot6}{3}=\frac{30}{3}=10}}</math> |
| + | |style="text-align:right;"|עוד הכינו הה' עם הו' שבטור השפל ונהיו ל' חלקנו אותם על הג' ונהיה החלק האחד י‫' | ||
|- | |- | ||
| | | | ||
| − | ::{| | + | ::Then, we divide the reserved 64 and an eighth by the 10; quotient is 6 integers, 2-fifths and one part of eighty. |
| + | :<math>\scriptstyle{\color{blue}{X=\frac{64+\frac{1}{8}}{10}=6+\frac{2}{5}+\frac{1}{80}}}</math> | ||
| + | |style="text-align:right;"|אחר כן חלקנו הס"ד ושמינית השמורים על הי' ונהיה החלק הא' ו' שלמים וב' חמישיות וחלק א' משמנים | ||
|- | |- | ||
| − | | | + | | |
| − | + | *Another example of the first proportional pentad, the problem is: if 2-quarters of a dinar in 4-eighths of a day earn 3-quarters of a dinar in 4-eighths of a day, how much will 4-quarters earn? | |
| − | + | |style="text-align:right;"|<big>דמיון</big> אחר על בעלת חמש צורות הראשונות אשר השאלה היא אם ב' רביעיות דינר בד' שמיניות היום הרויחו ג' רביעיות דינר בד' שמיניות היום כמה ירויחו ד' רביעיות | |
| − | |||
| − | |||
| − | |||
| − | | | ||
|- | |- | ||
| | | | ||
| − | + | :We write them according to the first rule like this: | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכתבנום כמשפט הראשון ככה |
|- | |- | ||
| | | | ||
| − | ::We | + | ::We multiply the 2 by the 4 in the upper line; it is 8. We keep it, because by this eight we divide the product of the numbers left in the upper line. |
| − | ::<math>\scriptstyle{\color{blue}{ | + | ::<math>\scriptstyle{\color{blue}{2\sdot4=8}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כפלנו הב' על הד' שבטור העליון ונהיו ח' ושמרנום כי על אלה השמנה נחלק כפל המספרים הנשארים אשר בטור העליון |
|- | |- | ||
| − | |||
| | | | ||
| + | ::Then, we multiply the 3 in the upper line by the 4 in the bottom line beneath it; it is 12. We multiply also the 12 by the 4 in the upper line next to it; it is 48. | ||
| + | ::<math>\scriptstyle{\color{blue}{3\sdot4\sdot4=12\sdot4=48}}</math> | ||
| + | |style="text-align:right;"|אחר כן כפלנו הג' שבטור העליון על הד' שבטור השפל אשר תחתיו ונהיה י"ב עוד הכינו הי"ב עם הד' שבטור העליון אשר בצדם ונהיה מ"ח | ||
|- | |- | ||
| | | | ||
| − | : | + | ::We divide it by the reserved 8; the quotient is six. We keep it. |
| − | : | + | ::<math>\scriptstyle{\color{blue}{\frac{48}{8}=6}}</math> |
| − | : | + | |style="text-align:right;"|חלקנו אותם על הח' השמורים ונהיה החלק הא' ששה ושמרנום |
| − | |style="text-align:right;"| | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | :: | + | ::We multiply also the 4 by the 8 in the bottom line; it is thirty-two. We keep it, because by this 32 we divide the product of the numbers left in the bottom line. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{4\sdot8=32}}</math> |
| + | |style="text-align:right;"|עוד כפלנו הד' על הח' שבטור השפל ונהיו שלשים ושנים ושמרנום כי על אלה הל"ב נחלק כפל המספרים הנשארים אשר בטור השפל | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::Then, we multiply the 4 by the 4 in the bottom line next to it; it is 16. We multiply also the 16 by the 8 next to it; it is 128. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{4\sdot4\sdot8=16\sdot8=128}}</math> |
| + | |style="text-align:right;"|‫[אח"כ כפלנו הד' על הד' אשר בטור השפל]‫<ref>P1031 om.</ref> בצדם ונהיו י"ו עוד כפלנו הי"ו עם [הח']‫<ref>P1031 הה'</ref> אשר בצדם ונהיו קכ"ח | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::We divide it by the reserved 32; the quotient is 4. |
| − | ::<math>\scriptstyle{\color{blue}{ | + | ::<math>\scriptstyle{\color{blue}{\frac{128}{32}=4}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|חלקנו אותם על הל"ב השמורים ונהיה החלק האחד ד‫' |
|- | |- | ||
| | | | ||
| − | : | + | :Then, we divide the six we kept by this 4; the quotient is 1 and a half. We know from this that the profit that would be earned by the 4-quarters of a dinar at 4-eighths of a day is one and a half dinar, if the 2-quarters at 4-eighths earned 3-quarters of a dinar. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{X=\frac{6}{4}=1+\frac{1}{2}}}</math> |
| + | |style="text-align:right;"|אחר כן חלקנו הששה אשר שמרנום על אלה הד' ונהיה החלק א' וחצי ומזה ידענו שהריוח אשר ירויחו הד' רביעיות דינר בד' שמיניות היום הוא אחד וחצי דינר אם הב' רביעיות בד' שמיניות הרויחו ג' רביעיות דינר | ||
|- | |- | ||
| + | !<span style=color:Green>Addition of Fractions</span> | ||
| | | | ||
| − | |||
|- | |- | ||
| − | | | + | |If you wish to sum up fractions with fractions: |
| − | + | |style="text-align:right;"|<big>ואם</big> רצית לקבץ שברים עם שברים | |
| − | |style="text-align:right;"| | ||
| − | |||
|- | |- | ||
| − | | | + | |You should extract their [common] denominator and see these fractions of the denominator, then relate them to the denominator and you will know how much is the sum. |
| − | + | |style="text-align:right;"|אתה צריך לעשות מורה מהם ולהביט השברים ההם על אותו המורה ולערוך אותם על המורה ואז תדע כמה יהיה הסך | |
|- | |- | ||
| | | | ||
| − | :: | + | *Example: if one asks: we summed 2 thirds with 3 quarters. How much are they? |
| − | + | :<math>\scriptstyle\frac{2}{3}+\frac{3}{4}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<big>דמיון</big> שאל שואל קבצנו ב' שלישיות עם ג' רביעיות כמה יהיו |
|- | |- | ||
| | | | ||
| − | ::We multiply | + | ::We multiply 3 by 4; it is 12 and this is the denominator. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{3\sdot4=12}}</math> |
| + | |style="text-align:right;"|הכינו הג' עם הד' ונהיו י"ב והוא המורה | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::Its 2-thirds are 8. |
| − | ::<math>\scriptstyle{\color{blue}{ | + | ::<math>\scriptstyle{\color{blue}{12\sdot\frac{2}{3}=8}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וב' שלישיותיו ח‫' |
|- | |- | ||
| | | | ||
| − | :: | + | ::Its 3-quarters are 9. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{12\sdot\frac{3}{4}=9}}</math> |
| + | |style="text-align:right;"|וג' רביעיותיו ט‫' | ||
|- | |- | ||
| | | | ||
| − | ::{| | + | :Their sum is 17. Relate it to the 12, which is a whole unit; it is one integer, a third, and one part of 12. |
| + | :<math>\scriptstyle{\color{blue}{\frac{2}{3}+\frac{3}{4}=\frac{8+9}{12}=\frac{17}{12}=1+\frac{1}{3}+\frac{1}{12}}}</math> | ||
| + | |style="text-align:right;"|ושניהם {{#annot:term|178,1841|Fg96}}מקובצים{{#annotend:Fg96}} י"ז תערכם אל הי"ב שהוא האחד השלם יהיו א' שלם ושליש וחלק א' מי"ב | ||
| + | |- | ||
| + | |If there are more types of fractions, do like this also. | ||
| + | |style="text-align:right;"|וכן אם היו מיני השברים יותר ככה תעשה | ||
|- | |- | ||
| − | | | + | | |
| − | + | *Example: we summed 2 thirds, 3 quarters and 4 fifths. How much is the sum? | |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle\frac{2}{3}+\frac{3}{4}+\frac{4}{5}</math> |
| − | + | |style="text-align:right;"|<big>דמיון</big> [קבצנו]‫<ref>P1031 om.</ref> ב' שלישיות וג' רביעיות וד' חמישיות כמה יהיה המקובץ | |
|- | |- | ||
| − | | | + | | |
| − | + | ::We multiply 3 by 4; it is 12. We multiply 12 by 5; it is 60 and this is the denominator. | |
| + | ::<math>\scriptstyle{\color{blue}{3\sdot4\sdot5=12\sdot5=60}}</math> | ||
| + | |style="text-align:right;"|כפלנו ג' על ד' ונהיו י"ב עוד כפלנו הי"ב על הה' ונהיו ס' והוא המורה | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::Its 2-thirds are 40. |
| − | ::<math>\scriptstyle{\color{blue}{40 | + | ::<math>\scriptstyle{\color{blue}{60\sdot\frac{2}{3}=40}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וב' שלישיותיו מ‫' |
|- | |- | ||
| | | | ||
| − | :: | + | ::Its 3-quarters are 45. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{60\sdot\frac{3}{4}=45}}</math> |
| + | |style="text-align:right;"|וג' רביעיותיו מ"ה | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::Its 4-fifths are 48. |
| − | ::<math>\scriptstyle{\color{blue}{ | + | ::<math>\scriptstyle{\color{blue}{60\sdot\frac{4}{5}=48}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וד' חמישיותיו מ"ח |
|- | |- | ||
| | | | ||
| − | : | + | :The total sum is 133. We relate it to 60, which is the denominator and it is the whole unit; the result is two integers, a fifth, and one part of sixty. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{\frac{2}{3}+\frac{3}{4}+\frac{4}{5}=\frac{40+45+48}{60}=\frac{133}{60}=2+\frac{1}{5}+\frac{1}{60}}}</math> |
| + | |style="text-align:right;"|{{#annot:term|178,1841|GFVv}}והכל מקובצים{{#annotend:GFVv}} קל"ג ערכנו אותם על ס' שהוא המורה והוא האחד השלם ונהיו שנים שלמים וחמישית אחת וחלק אחד מששים | ||
| + | |- | ||
| + | |Similarly, if you want to sum up more than these, proceed according to this rule. | ||
| + | |style="text-align:right;"|וכן אם תרצה לקבץ יותר מאלה כן תעשה כמשפט הזה | ||
|- | |- | ||
| + | !<span style=color:Green>Division of Fractions</span> | ||
| | | | ||
| − | |||
|- | |- | ||
| − | | | + | |If you wish to divide fractions by fractions: |
| − | + | |style="text-align:right;"|ואם תרצה לחלק נשברים על נשברים | |
| − | |style="text-align:right;"| | ||
| − | |||
|- | |- | ||
| − | | | + | |Look for one denominator for both and take from it the number of parts required, then divide them by each other and the result is the sought. |
| − | + | |style="text-align:right;"|בקש מורה אחד לשניהם וקח ממנו כמספר החלקים הדרושים וחלק זה על זה והעולה הוא המבוקש | |
|- | |- | ||
| | | | ||
| − | : | + | *Example: we wish to divide 4-ninths by 5-sevenths. |
| − | + | :<math>\scriptstyle\frac{4}{9}\div\frac{5}{7}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<big>דמיון</big> רצינו לחלק ד' תשיעיות על ה' שביעיות |
|- | |- | ||
| | | | ||
| − | :: | + | ::The denominator is 63, because 9 times 7 is 63. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{9\sdot7=63}}</math> |
| + | |style="text-align:right;"|והמורה הוא ס"ג כי ט' פעמים ז' הם ס"ג | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::Its 4-ninths are 28. |
| − | ::<math>\scriptstyle{\color{blue}{ | + | ::<math>\scriptstyle{\color{blue}{63\sdot\frac{4}{9}=28}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וד' תשיעיותיו הם כ"ח |
|- | |- | ||
| | | | ||
| − | :: | + | ::Its 5-sevenths are 45. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{63\sdot\frac{5}{7}=45}}</math> |
| + | |style="text-align:right;"|וה' שביעיותיו הם מ"ה | ||
|- | |- | ||
| | | | ||
| − | ::{| | + | :We divide the 28 by the 45; the result is one half, a ninth, and one part of ninety; and this is the required. |
| + | :<math>\scriptstyle{\color{blue}{\frac{4}{9}\div\frac{5}{7}=\frac{28}{45}=\frac{1}{2}+\frac{1}{9}+\frac{1}{90}}}</math> | ||
| + | |style="text-align:right;"|חלקנו הכ"ח על המ"ה ונהיו חצי אחד ותשיעית אחד וחלק א' מתשעים וזהו המבוקש | ||
| + | |- | ||
| + | |If there are integers and fractions, do as follows: | ||
| + | |style="text-align:right;"|ואם היו שם שלמים עם שברים תעשה ככה | ||
|- | |- | ||
| − | | | + | |Seek for the denominator, which is the one unit, look at the [integer] and multiply it by it, add the fractions to it; do the same with the number, by which you divide; then divide by each other and the result is the required. |
| − | + | |style="text-align:right;"|בקש המורה והוא הא' השלם ועליו תביט את מספר החלקים ותכפלם כמספרו ותחבר גם את השברים עמהם וכן תעשה גם במספר אשר תחלוק עליו אחר ‫<ref>39r</ref>כן חלק זה על זה והעולה הוא המבוקש | |
| − | |style="text-align:right;"| | ||
| − | |||
|- | |- | ||
| − | | | + | | |
| − | + | *Example: we wish to divide 3 integers and 2-fifths by 2 integers and 4-sevenths. | |
| + | :<math>\scriptstyle\left(3+\frac{2}{5}\right)\div\left(2+\frac{4}{7}\right)</math> | ||
| + | |style="text-align:right;"|<big>דמיון</big> רצינו לחלק ג' שלמים וב' חמישיות על ב' שלמים וד' שביעיות אחד | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::The denominator is 35, because 5 times 7 is 35 and it is the whole. |
| − | ::<math>\scriptstyle{\color{blue}{ | + | ::<math>\scriptstyle{\color{blue}{5\sdot7=35}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והמורה ל"ה כי ה' פעמים ז' הם ל"ה והוא הא' השלם |
|- | |- | ||
| | | | ||
| − | ::We | + | ::The 3 integers are 105, because 3 times 35 is 105; the 2-fifths are 14, because a fifth of the 35 is 7. We add them to the 105; it is 119. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\left(35\sdot3\right)+\left(35\sdot\frac{2}{5}\right)=105+14=119}}</math> |
| + | |style="text-align:right;"|והג' שלמים הם ק"ה כי ג' פעמים ל"ה הם ק"ה והב' חמישיות הם י"ד כי חמישית הל"ה הם ז' חברנום עם הק"ה ונהיו קי"ט | ||
|- | |- | ||
| | | | ||
| − | ::We | + | ::We also set the 2 other integers as 70, and the 4-sevenths as 20, because a seventh of the 35 is 5. We add them to the 70; it is 90. |
| − | ::<math>\scriptstyle{\color{blue}{ | + | ::<math>\scriptstyle{\color{blue}{\left(35\sdot2\right)+\left(35\sdot\frac{4}{7}\right)=70+20=90}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|עוד שמנו הב' שלמים האחרים ע' והד' שביעיות כ' כי שביעית הל"ה הם ה' חברנום עם הע' ונהיו צ‫' |
|- | |- | ||
| | | | ||
| − | + | :We divide the 119 by it; the result is one integer and 29 parts remain, which are 2-ninths of the 90 and one tenth. | |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{\left(3+\frac{2}{5}\right)\div\left(2+\frac{4}{7}\right)=\frac{119}{90}=1+\frac{29}{90}=1+\frac{2}{9}+\frac{1}{10}}}</math> |
| + | |style="text-align:right;"|חלקנו עליהם הקי"ט ונהיה אחד שלם ונשארו כ"ט חלקים שהם ב' תשיעיות מן הצ' ועשירית אחד | ||
|- | |- | ||
| + | !<span style=color:Green>Subtraction of Fractions</span> | ||
| | | | ||
| − | |||
|- | |- | ||
| − | | | + | |Similarly, if you wish to subtract fractions from fractions, do as follows: |
| − | + | |style="text-align:right;"|וכן אם רצית לחסר שברים משברים ככה תעשה | |
| − | |style="text-align:right;"| | + | |- |
| − | + | |First, seek for the denominator and see its parts, then subtract those from those. | |
| + | |style="text-align:right;"|בקש המורה בתחלה וראה חלקיו וחסר אלה מאלה | ||
|- | |- | ||
| − | | | + | | |
| − | + | *Example: we wish to subtract 2-sevenths from 4-ninths. | |
| + | :<math>\scriptstyle\frac{4}{9}-\frac{2}{7}</math> | ||
| + | |style="text-align:right;"|<big>דמיון</big> רצינו לחסר ב' שביעיות מד' תשיעיות | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::The denominator is 63. |
| − | + | |style="text-align:right;"|והמורה הוא ס"ג | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::Its 2-sevenths are 18. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{63\sdot\frac{2}{7}=18}}</math> |
| + | |style="text-align:right;"|וב' שביעיותיו י"ח | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::Its 4-ninths are 28. |
| − | ::<math>\scriptstyle{\color{blue}{ | + | ::<math>\scriptstyle{\color{blue}{63\sdot\frac{4}{9}=28}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וארבע תשיעיותיו כ"ח |
|- | |- | ||
| | | | ||
| − | : | + | :Subtract 18 from 28; 10 remains, which is a little less than a sixth. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{\frac{4}{9}-\frac{2}{7}=\frac{28-18}{63}=\frac{10}{63}<\frac{1}{6}}}</math> |
| + | |style="text-align:right;"|תחסר י"ח מכ"ח ונשארו י' שהם פחות מששית מעט | ||
| + | |- | ||
| + | |Now, I will train you in the issue of the problems, so that you will be quick in your calculation. | ||
| + | |style="text-align:right;"|ועתה ארגילך בענין השאלות כדי שתהיה זריז בחשבונך | ||
|} | |} | ||
| − | |||
{| | {| | ||
|- | |- | ||
| | | | ||
| − | + | ||
| − | + | === The Place of the Problems === | |
| + | |||
| + | !style="width:45%; text-align:right;"|<big>מקום השאלות</big> | ||
|- | |- | ||
| + | !<span style=color:Green>Multiplication of Fractions</span> | ||
| | | | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | :: | + | :{{#annot:⁹/₁₃×¹⁷/₁₉|17|v4BY}}1) How much are 9 parts of 13 multiplied by 17 parts of 19? |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle\frac{9}{13}\times\frac{17}{19}</math> |
| + | |style="text-align:right;"|<big>א</big> כמה ט' חלקים מי"ג הכפולים על י"ז חלקים מי"ט{{#annotend:v4BY}} | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::These do not have an expressible ratio, therefore you should know how to multiply and what to do. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כי אלה אין להם ערך מדובר לכן ראוי שתדע איך תכפול ומה תעשה |
|- | |- | ||
| | | | ||
| − | :: | + | ::<span style=color:Green>common denominator:</span> We should know the denominator first. We multiply 13 by 19; it is 247 and this is the denominator. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{13\sdot19=247}}</math> |
| + | |style="text-align:right;"|ראוי שנדע בתחלה המורה והנה נכפול י"ג על י"ט יהיו רמ"ז והוא המורה | ||
|- | |- | ||
| − | |||
| | | | ||
| + | ::Then, we take 19 for each of the 9 parts; it is 171 and this is the first number. | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{9}{13}\sdot19=\frac{171}{13}}}</math> | ||
| + | |style="text-align:right;"|אחר כן נקח לכל א' מט' חלקים י"ט נהיו קע"א והוא המספר האחד | ||
|- | |- | ||
| | | | ||
| − | : | + | ::We take also 13 for each of the 17 parts; it is 221. |
| − | + | ::<math>\scriptstyle{\color{blue}{\frac{17}{19}\sdot13=\frac{221}{19}}}</math> | |
| − | : | + | |style="text-align:right;"|עוד נקח לכל א' מי"ז חלקים י"ג נהיו רכ"א |
| − | : | ||
| − | |||
| − | |||
| − | |||
| − | |style="text-align:right;"| | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | :: | + | ::We multiply one by the other; it is 37791. |
| − | + | |style="text-align:right;"|נכפול זה על זה נהיו ל"ז אלפים תשצ"א | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::We divide it by 247; the result is 153. |
| − | ::<math>\scriptstyle{\color{blue}{ | + | ::<math>\scriptstyle{\color{blue}{\frac{171}{13}\sdot\frac{221}{19}=\frac{37791}{247}=153}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|חלקנו אותם על רמ"ז ועלו קנ"ג |
|- | |- | ||
| | | | ||
| − | :: | + | ::The product of 9 by 17 is also 153. |
| − | ::<math>\scriptstyle{\color{blue}{\ | + | ::<math>\scriptstyle{\color{blue}{9\sdot17=153}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והנה העולה מכפל ט' בי"ז גם כן קנ"ג |
|- | |- | ||
| | | | ||
| − | : | + | ::The ratio of it to 247 is as the ratio of the first number to the square of 247 and this is what we sought. |
| − | ::<math>\scriptstyle{\color{blue}{ | + | ::<math>\scriptstyle{\color{blue}{153:247=37791:247^2}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וערך זה אל רמ"ז כערך המספר הראשון אל מרובע רמ"ז וזהו מה שבקשנו |
|- | |- | ||
| | | | ||
| − | ::If you want to | + | ::If you want to be precise, divide 153 by 19; the result is 8 parts of 13 and one fraction remains, which is one part of 19. |
| − | |style="text-align:right;"|ואם תרצה | + | ::<math>\scriptstyle{\color{blue}{\frac{9}{13}\times\frac{17}{19}=\frac{\frac{153}{19}}{13}=\frac{8}{13}+\frac{\frac{1}{19}}{13}}}</math> |
| + | |style="text-align:right;"|ואם תרצה לדקדקו חלק קנ"ג על י"ט יהיה העולה [ח']‫<ref>P1031 ז'</ref> חלקים מי"ג וישאר [שבר א' שהוא שבר חלק]‫<ref>P1031 ב' שברים</ref> מי"ט | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::Or, if you want, we divide 153 by 13; the result is 11 parts of 19 and 10 parts of 13 of one part of 19. |
| − | + | ::<math>\scriptstyle{\color{blue}{\frac{9}{13}\times\frac{17}{19}=\frac{\frac{153}{13}}{19}=\frac{11}{19}+\frac{\frac{10}{13}}{19}}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|או אם תרצה חלקנו הקנ"ג על י"ג ויהיו י"א חלקים שלמים מי"ט וי' שברים שי"ג מהם עושה חלק א' מי"ט |
|- | |- | ||
| + | !<span style=color:Green>How Much Problems</span> | ||
| | | | ||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| + | !<span style=color:Green>Road</span> | ||
| | | | ||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | :: | + | :{{#annot:road|648|ZjtJ}}2) Question: a road, we add to it its quarter and its fifth and the sum is 10 miles. |
| − | : | + | :How long is the road? |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle X+\frac{1}{4}X+\frac{1}{5}X=10</math> |
| + | |style="text-align:right;"|<big>ב</big> <big>שאלה</big> דרך חברנו אליה רביעיתה וחמישיתה והיתה י' מילין<br> | ||
| + | כמה הדרך{{#annotend:ZjtJ}} | ||
|- | |- | ||
| | | | ||
| − | ::We | + | ::<span style=color:Green>False Position - common denominator:</span> We define the denominator twenty, because 4 times 5 is 20. |
| − | ::<math>\scriptstyle{\color{blue}{ | + | ::<math>\scriptstyle{\color{blue}{4\sdot5=20}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|נשים המורה עשרים כי ד' פעמים ה' הם כ‫' |
|- | |- | ||
| − | |||
| | | | ||
| + | ::Its quarter and its fifth are 9. We add it to the denominator; it is 29. | ||
| + | ::<math>\scriptstyle{\color{blue}{20+\left(\frac{1}{4}\sdot20\right)+\left(\frac{1}{5}\sdot20\right)=29}}</math> | ||
| + | |style="text-align:right;"|ורביעיתן וחמישיתן הן ט' נחברם עם המורה נהיו כ"ט | ||
|- | |- | ||
| − | |||
| | | | ||
| + | ::<span style=color:Green>Rule of Three:</span> We multiply 20 by 10; it is 200. | ||
| + | |style="text-align:right;"|נכפול הכ' עם י' ונהיו ר‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | ::We divide it by 29; it is 6 miles, half a mile, a third of a mile, and approximately one part of 15 and this is [the length of] the road. |
| − | + | ::<math>\scriptstyle{\color{blue}{X=\frac{20\sdot10}{29}=\frac{200}{29}\approx6+\frac{1}{2}+\frac{1}{3}+\frac{1}{15}}}</math> | |
| − | : | + | |style="text-align:right;"|נחלקם על כ"ט ונהיו ו' מילין וחצי מיל ושליש מיל וחלק א' [מט"ו]‫<ref>P1031 מה'</ref> בקירוב וככה היא הדרך |
| − | |style="text-align:right;"| | ||
| − | |||
| − | |||
|- | |- | ||
| + | !<span style=color:Green>Money</span> | ||
| | | | ||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :{{#annot:money|648|PsiN}}3) Question: we take a ninth of an amount of money, its tenth, and its one part of 11. How much is the ratio of all of this to the amount of money? |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle\left(\frac{1}{9}X+\frac{1}{10}X+\frac{1}{11}X\right):X</math> |
| + | |style="text-align:right;"|<big>ג</big> <big>שאלה</big> לקחנו תשיעית ממון ועשיריתו וחלק א' מי"א ממנו<br> | ||
| + | כמה הוא כל זה מערך הממון{{#annotend:PsiN}} | ||
|- | |- | ||
| | | | ||
| − | + | :This is easy for the one who knows to add fractions, as we wrote concerning their addition. | |
| − | + | |style="text-align:right;"|והנה זה נקל הוא מי שידע לחבר השברים כאשר כתבנו בחבורם | |
| − | |||
| − | |||
| − | |style="text-align:right;"| | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | ::We | + | ::We do as follows: we look for a denominator by multiplying nine by ten; it is 90. Then 90 by 11; it is 990 and this is the denominator. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{9\sdot10\sdot11=90\sdot11=990}}</math> |
| + | |style="text-align:right;"|וכן נעשה נבקש מורה והוא שנכפול תשעה על עשרה ונהיו צ' עוד צ' על י"א ונהיו תתק"צ וזהו המורה | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::Its ninth is 110; its tenth is 99; its one part of 11 is 90; and the total sum is 299. |
| − | ::<math>\scriptstyle{\color{blue}{ | + | |style="text-align:right;"|ותשיעיתו ק"י ועשריתו צ"ט וחלק אחד עשרה ממנו צ' והכל מחוברים רצ"ט |
| − | + | |- | |
| + | |colspan=2| | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(\frac{1}{9}\sdot990\right)+\left(\frac{1}{10}\sdot990\right)+\left(\frac{1}{11}\sdot990\right)=110+99+90=299}}</math> | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::The ratio of 299 to 990 is the ratio of these to the amount of money. |
| − | ::<math>\scriptstyle{\color{blue}{ | + | ::<math>\scriptstyle{\color{blue}{299:990=\left(\frac{1}{9}X+\frac{1}{10}X+\frac{1}{11}X\right):X}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וערך רצ"ט אל תתק"צ ככה ערך אלה אל הממון |
|- | |- | ||
| | | | ||
| − | :: | + | ::We divide it by 90, which is one part of 11; it is 3 parts of 11 and 29 parts of 90, which are one-third of one part of 11 minus something. |
| − | ::<math>\scriptstyle{\color{blue}{ | + | ::<math>\scriptstyle{\color{blue}{299:990=\frac{\frac{299}{90}}{11}=\frac{3}{11}+\frac{\frac{29}{90}}{11}=\frac{3}{11}+\frac{\frac{1}{3}}{11}-\ldots}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|חלקנו אותם אל צ' שהוא חלק אחד מי"א ונהיו ג' חלקים מי"א וכ"ט חלקים מצ' שהם שלישית חלק מי"א פחות משהו |
|- | |- | ||
| | | | ||
| − | ::{| | + | :{{#annot:money|648|1vbG}}4) Question: an amount of money, we add to it its half, its third, its fifth, and its sixth and the sum is sixty. |
| + | :How much is the amount of money? | ||
| + | :<math>\scriptstyle X+\frac{1}{2}X+\frac{1}{3}X+\frac{1}{5}X+\frac{1}{6}X=60</math> | ||
| + | |style="text-align:right;"|<big>ד</big> <big>שאלה</big> ממון הוספנו עליו מחציתו שלישיתו חמישיתו וששיתו ובין הכל היו ששים<br> | ||
| + | כמה היה הממון{{#annotend:1vbG}} | ||
|- | |- | ||
| − | | | + | | |
| − | + | ::We know that the half, the third, and the six are one integer. | |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\frac{1}{2}+\frac{1}{3}+\frac{1}{6}=1}}</math> |
| − | + | |style="text-align:right;"|ידענו שהחצי והשלישית והששית הוא אחד שלם | |
|- | |- | ||
| − | | | + | | |
| − | | | + | ::We think as if it were one, so it is now two and a fifth. |
| + | ::<span style=color:Green>False Position:</span> <math>\scriptstyle{\color{blue}{1+\frac{1}{2}+\frac{1}{3}+\frac{1}{5}+\frac{1}{6}=1+1+\frac{1}{5}=2+\frac{1}{5}}}</math> | ||
| + | |style="text-align:right;"|ונחשב שהיה לו אחד ויש לו עתה שנים וחמשיתן | ||
|- | |- | ||
| | | | ||
| − | ::We | + | ::<span style=color:Green>Rule of Three:</span> We divide the sixty by 2 and a fifth and so is the amount of money. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|נחלק הששים על ב' והחמישית וכן היה הממון |
|- | |- | ||
| | | | ||
| − | :: | + | ::Convert the 2 integers into fifths, since we have a fifth, then we add the fifth that we have to them; they are 11 fifths. |
| − | + | |style="text-align:right;"|והנה תשיב הב' [שלימי']‫<ref>P1031 שלישיות</ref> אל חמישיות מפני שיש לנו חמישית ונחבר גם החמישית שיש לנו עמם ונהיו י"א חמישיות | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| − | |||
| | | | ||
| + | ::We multiply 5 by 60; it is 300 and this is the denominator. | ||
| + | |style="text-align:right;"|‫[נכפול]‫<ref>P1031 בכפל</ref> ה' על ס' ונהיו ש' והוא המורה | ||
|- | |- | ||
| | | | ||
| − | : | + | ::We divide it by 11; the quotient is 27 and three parts of 11 and this is the amount of money. |
| − | + | ::<math>\scriptstyle{\color{blue}{X=\frac{60}{2+\frac{1}{5}}=\frac{60}{\frac{11}{5}}=\frac{60\sdot5}{11}=\frac{300}{11}=27+\frac{3}{11}}}</math> | |
| − | + | |style="text-align:right;"|נחלקם על י"א והיה החלק כ"ז ושלשה חלקים מי"א וככה היה הממון | |
| − | |||
| − | |||
| − | : | ||
| − | |||
| − | :<math>\scriptstyle\ | ||
| − | |style="text-align:right;"| | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| + | !<span style=color:Green>How Many Problem - horses</span> | ||
| | | | ||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | :: | + | :{{#annot:horses|649|nuR5}}5) Question: a riding man saw a man pulling horses and said to him: where are you leading these 100 horses? |
| − | ::<math>\scriptstyle | + | :He answered him: these and other like them and a half of them and a quarter of them with the horse you ride are a hundred. |
| − | |style="text-align:right;"| | + | :How much were the horses? |
| + | :<math>\scriptstyle X+X+\frac{1}{2}X+\frac{1}{4}X+1=100</math> | ||
| + | |style="text-align:right;"|<big>ה</big> <big>שאלה</big> אדם רוכב ראה איש מושך סוסים ואמר לו אנה אתה מוליך אלה הק' סוסים<br> | ||
| + | והשיב לו אלה ואחרים כמותם ומחציתם ורביעיתם ועם הסוס שאתה רוכב הם מאה<br> | ||
| + | כמה היו הסוסים{{#annotend:nuR5}} | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::We subtract the rider's horse; 99 remains. |
| − | ::<math>\scriptstyle{\color{blue}{ | + | ::<math>\scriptstyle{\color{blue}{100-1=99}}</math> |
| − | |style="text-align:right;"|הנה | + | |style="text-align:right;"|הנה נוציא הסוס שרוכב זה ונשארו צ"ט |
|- | |- | ||
| | | | ||
| − | ::We | + | ::<span style=color:Green>False Position:</span> We have two, a half, and a quarter. Since we have a quarter, we convert all into quarters: the 2 integers are 8 quarters; the half is 2; so they are 10. We add the quarters that we have to them also; they are 11 quarters. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{1+\frac{1}{2}+\frac{1}{4}=\frac{8}{4}+\frac{2}{4}+\frac{1}{4}=\frac{11}{4}}}</math> |
| + | |style="text-align:right;"|יש לנו שנים ומחציתו ורביעית ומפני ‫<ref>39v</ref>שיש לנו רביעית נשיב הכל לרביעיות והנה הב' שלמים ח' רביעיות והמחצית ב' הרי י' נחבר גם הרביעיות שיש לנו עמם נהיו י"א רביעיות | ||
|- | |- | ||
| | | | ||
| − | :::We | + | ::<span style=color:Green>Rule of Three:</span> We return them into the ratios and write: if the 11-quarters yield 99, how much will the 4-quarter that are one integer [yield]? |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\frac{11}{4}:99=\frac{4}{4}:x}}</math> |
| + | |style="text-align:right;"|אחר נשיב אותם אל הערכים ונכתוב אם הי"א רביעיות נתנו צ"ט הד' רביעיות שהוא האחד השלם כמה | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::We multiply the means, which are 4 by 99, then divide them by 11, which is the extreme; it is 36 and this is the number of the horses. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{X=\frac{4\sdot99}{11}=36}}</math> |
| + | |style="text-align:right;"|נכפול האמצעיים שהם הד' על הצ"ט ונחלקם על י"א שהוא הקצה ונהיו ל"ו ככה מספר הסוסים | ||
|- | |- | ||
| + | !<span style=color:Green>Buy and Sell Problems</span> | ||
| | | | ||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | :: | + | :{{#annot:WP|641|ZPuq}}6) Question: a man bought 24 liṭra for 24 dinar. He sold 12 [of them] at a liṭra and a quarter of a liṭra for one dinar, and the [other] 12 liṭra at a liṭra minus a quarter of a liṭra for one dinar. |
| − | |style="text-align:right;"| | + | :We want to know: did he earn or lose? |
| + | :<math>\scriptstyle\left(\frac{12}{1+\frac{1}{4}}+\frac{12}{1-\frac{1}{4}}\right)-24</math> | ||
| + | |style="text-align:right;"|<big>ו</big> <big>שאלה</big> אדם קנה כ"ד ליטרא בכ"ד דינר ומכר הי"ב ליטרא ורביע ליטרא בדינר והי"ב ליטרין מכר ליטרא פחות רביע ליטרא בדינר<br> | ||
| + | נבקש לדעת אם הרויח או הפסיד{{#annotend:ZPuq}} | ||
|- | |- | ||
| | | | ||
| − | ::We | + | ::We convert the first 12 liṭra into quarters; they are 48 |
| − | + | |style="text-align:right;"|נשיב הי"ב ליטרא הראשונים לרביעיות ונהיו מ"ח | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::We divide them by 5, since he sold 5 quarters for one dinar; it is 9, a hald, and a tenth, and these are the dinar he earned from the first 12 liṭra. |
| + | |style="text-align:right;"|נחלקם על ה' כי ה' רביעיות מכר [בדינר]‫<ref>P1031 om.</ref> ונהיו ט' וחצי ועשירית ואלה הם הדינרים שלקח מהי"ב ליטרא הראשונים | ||
|- | |- | ||
| − | | | + | | |
| − | + | ::We multiply the other 12 liṭra by 4, since he sold a liṭra minus a quarter of a liṭra, which is 3-quarters, for one dinar; they are 48. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|עוד נכה הי"ב ליטרא [האחרוני']‫<ref>P1031 om.</ref> בד' כי ליטרא פחות רביע הם ג' רביעיות מכר בדינר ונהיו מ"ח |
| − | |||
|- | |- | ||
| − | | | + | | |
| − | | | + | ::We divide them by 3; it is 16, and these are the dinar he earned from the other 12 liṭra. |
| + | |style="text-align:right;"|לכן נחלקם על ג' ונהיו י"ו ואלה הם הדינרים שלקח מהי"ב ליטרא האחרונים | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::We sum up the two amounts of money; they are 25, a half, and a tenth. |
| − | + | |style="text-align:right;"|נחבר שני [הממונות]‫<ref>P1031 המדרגות</ref> נהיו כ"ה וחצי ועשירית | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::We subtract the 24 dinar of the original amount; dinar, a half, and a tenth remain, and this is the profit. |
| + | |style="text-align:right;"|נוציא כ"ד דינר של הקרן נשאר דינר וחצי ועשירית וזהו הריוח | ||
|- | |- | ||
| − | | | + | |colspan=2| |
| − | + | ::<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle\left(\frac{12}{1+\frac{1}{4}}+\frac{12}{1-\frac{1}{4}}\right)-24&\scriptstyle=\left(\frac{4\sdot12}{5}+\frac{4\sdot12}{3}\right)-24=\left(\frac{48}{5}+\frac{48}{3}\right)-24=\left[\left(9+\frac{1}{2}+\frac{1}{10}\right)+16\right]-24\\&\scriptstyle=\left(25+\frac{1}{2}+\frac{1}{10}\right)-24=1+\frac{1}{2}+\frac{1}{10}\\\end{align}}}</math> | |
| − | |||
| − | { | ||
|- | |- | ||
| − | | | + | | |
| − | + | :{{#annot:WP|641|NJEy}}7) Question: a man bought three fifths of a liṭra for one pašuṭ, then he sold four sevenths of a liṭra for one pašuṭ and he earned one pašuṭ.<br> | |
| + | :How much money did he have originally? | ||
| + | :<math>\scriptstyle\frac{\frac{3}{5}X}{\frac{4}{7}}=X+1</math> | ||
| + | |style="text-align:right;"|<big>ז</big> <big>שאלה</big> אדם קנה ג' חמישיות ליטרא בפשוט ומכר ד' שביעיות ליטרא בפשוט והרויח פשוט<br> | ||
| + | כמה היה ממונו{{#annotend:NJEy}} | ||
|- | |- | ||
| | | | ||
| − | ::We | + | ::<span style=color:Green>common denominator:</span> We look for a denominator; it is 35. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|נבקש המורה והוא ל"ה |
|- | |- | ||
| | | | ||
| − | :: | + | ::Its 3-fifths are 21. |
| − | ::<math>\scriptstyle{\color{blue}{ | + | ::<math>\scriptstyle{\color{blue}{x+1=\frac{3}{5}\sdot35=21}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וג' חמישיותיו כ"א |
|- | |- | ||
| | | | ||
| − | :: | + | ::Its 4-sevenths are 20. |
| − | ::<math>\scriptstyle{\color{blue}{ | + | ::<math>\scriptstyle{\color{blue}{x=\frac{4}{7}\sdot35=20}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וד' שביעיותיו כ‫' |
|- | |- | ||
| | | | ||
| − | :: | + | :*<span style=color:Green>Check:</span> The amount of money was 20 pešuṭim, because for each pašuṭ he earns one part of 20 of a pašuṭ and when he sells for 20 pešuṭim he earns one pašuṭ. |
| + | |style="text-align:right;"|והממון היה כ' פשוטים כי בכל פשוט ירויח חלק אחד מכ' בפשוט וכשימכור של כ' פשוטים ירויח פשוט אחד | ||
|- | |- | ||
| − | | | + | | |
| − | + | :{{#annot:WP|641|226G}}Question: a man bought 9 parts of 17 parts of a liṭra for one pašuṭ, then he sold 10 parts of 19 parts [of a liṭra] for one pašuṭ and he earned one pašuṭ. | |
| − | |style="text-align:right;"| | + | :How much was the money? |
| − | { | + | :<math>\scriptstyle\frac{\frac{9}{17}X}{\frac{10}{19}}=X+1</math> |
| + | |style="text-align:right;"|<big>שאלה</big> אדם קנה ט' חלקים מי"ז חלקי ליטרא בפשוט ומכר י' חלקים מי"ט בפשו' והרויח פשוט<br> | ||
| + | כמה היה הממון{{#annotend:226G}} | ||
|- | |- | ||
| − | | | + | | |
| − | + | ::<span style=color:Green>common denominator:</span> We look for a denominator by multiplying 17 by 19; it is 323. | |
| + | ::<math>\scriptstyle{\color{blue}{17\sdot19=323}}</math> | ||
| + | |style="text-align:right;"|נבקש המורה והוא שנכפול י"ז על י"ט ונהיו שכ"ג | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::It is known that 9 parts of 17 are 171 and this was the amount of money. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{x+1=\frac{9}{17}\sdot323=171}}</math> |
| + | |style="text-align:right;"|כי ידוע כי ט' חלקים מי"ז הם קע"א וככה היה הממון | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::10 parts of 19 are 170. |
| − | ::<math>\scriptstyle{\color{blue}{ | + | ::<math>\scriptstyle{\color{blue}{x=\frac{10}{19}\sdot323=170}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|‫[וי']‫<ref>P1031 וי"ח</ref> חלקים מי"ט הם ק"ע |
|- | |- | ||
| | | | ||
| − | :*<span style=color:Green>Check:</span> | + | :*<span style=color:Green>Check:</span> So, he earned one pašuṭ. |
| − | + | |style="text-align:right;"|והנה הרויח פשוט | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| + | !<span style=color:Green>How Much Problem - Money</span> | ||
| | | | ||
| − | |||
|- | |- | ||
| − | |||
| | | | ||
| + | :{{#annot:money|648|GeZy}}8) Question: an amount of money, we sum its third, its quarter, and its fifth and the sum is thirty. | ||
| + | :How much is the amount of money? | ||
| + | :<math>\scriptstyle\frac{1}{3}X+\frac{1}{4}X+\frac{1}{5}X=30</math> | ||
| + | |style="text-align:right;"|<big>ח</big> <big>שאלה</big> ממון חברנו שלישיתו רביעיתו חמישיתו והיו שלשים<br> | ||
| + | כמה הוא כל הממון{{#annotend:GeZy}} | ||
|- | |- | ||
| − | | | + | | |
| − | : | + | ::<span style=color:Green>False Position - common denominator:</span> We look for the denominator; it is sixty. |
| − | + | ::<math>\scriptstyle{\color{blue}{60}}</math> | |
| − | :<math>\scriptstyle | + | |style="text-align:right;"|נבקש המורה והוא ששים |
| − | |style="text-align:right;"| | ||
| − | |||
|- | |- | ||
| | | | ||
| − | :: | + | ::Its third is 20; its quarter is 15; its fifth is 12; together they are 47. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\left(\frac{1}{3}\sdot60\right)+\left(\frac{1}{4}\sdot60\right)+\left(\frac{1}{5}\sdot60\right)=20+15+12=47}}</math> |
| − | + | |style="text-align:right;"|ושלישיתו כ' ורביעיתו ט"ו וחמישיתו י"ב וכלם מ"ז | |
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{X | + | ::<span style=color:Green>Rule of Three:</span> As the ratio of 47 to sixty so is the ratio of thirty to the whole amount of money. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{47:60=30:X}}</math> |
| + | |style="text-align:right;"|הנה כערך מ"ז אל ששים כן ערך שלשים אל כל הממון | ||
|- | |- | ||
| | | | ||
| − | : | + | ::We write like this: if 47 yields 60, how much will thirty yield? |
| − | : | + | |style="text-align:right;"|ונכתוב ככה אם המ"ז יתנו ס' השלשים מה יתנו |
| − | |||
| − | |||
| − | |style="text-align:right;"|' | ||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | :: | + | ::{|class="wikitable" style="text-align:right; color:blue" |
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |0||30||60||47 |
| − | + | |} | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"| |
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:right;" | ||
|- | |- | ||
| − | | | + | |0||03||06||74 |
| − | + | |} | |
| − | |||
|- | |- | ||
| − | |||
| | | | ||
| − | + | ::We miltiply the means; it is 1800. | |
| − | + | |style="text-align:right;"|נכפול האמצעיים ונהיו אלף ת"ת | |
| − | : | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |style="text-align:right;"| | ||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | :: | + | ::We divide it by the extreme, which is 47; the quotient is 38, a quarter, and 2 parts and a quarter of a part of 47. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{X=\frac{30\sdot60}{47}=\frac{1800}{47}=38+\frac{1}{4}+\frac{2+\frac{1}{4}}{47}}}</math> |
| + | |style="text-align:right;"|נחלקם על הקצה שהוא המ"ז נהיה החלק ל"ח ורביעית וב' חלקי' ורביע חלק ממ"ז | ||
|- | |- | ||
| + | !<span style=color:Green>Partnership Problem - For the Same Time</span> | ||
| | | | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :{{#annot:five partners|661|C96F}}9) Question: five people - the first gave 6 dinar, the second 7 dinar, the third 8 dinar, the fourth 9 dinar, and the fifth 10 dinar. |
| − | :: | + | :They earned a total of 11 dinar. |
| − | |style="text-align:right;"| | + | :How much should each of them take [from the profit]? |
| + | |style="text-align:right;"|<big>ט</big> <big>שאלה</big> אם אנשים היו חמשה נתן הא' ו' דינרי' והשני ז' דינרים והשלישי ח' דינרים והרביעי ט' דינרים והחמישי י' דינרים<br> | ||
| + | והרויחו בין הכל י"א דינרים<br> | ||
| + | כמה יקח כל א' מהם{{#annotend:C96F}} | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::The 11 dinar is what is earned by the sum of all these dinar. |
| − | + | |style="text-align:right;"|הנה אלה הי"א דינרי' הרויחו אותם כל הדינרים מקובצים | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::We look how much they are, then we convert them to the ratio of each and as the ratio of the money of each so each takes. |
| − | + | |style="text-align:right;"|ונראה אותם כמה הם אחר כן נשיב אותם אל הערך של כל אחד ואחד וכפי ערך ממונו ככה יקח | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::The sum of the given dinar is 40 dinar. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{6+7+8+9+10=40}}</math> |
| + | |style="text-align:right;"|והנה הדינרים מקובצים הם מ' דינר | ||
|- | |- | ||
| | | | ||
| − | ::the | + | ::We write the ratio of the first as follows: |
| − | + | |style="text-align:right;"|ונכתוב הערך של הראשון ככה | |
| − | |||
| − | |||
| − | |||
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
::{|class="wikitable" style="text-align:right; color:blue" | ::{|class="wikitable" style="text-align:right; color:blue" | ||
|- | |- | ||
| − | |0|| | + | |0||6||11||40 |
|} | |} | ||
|style="text-align:right;"| | |style="text-align:right;"| | ||
{|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:right;" | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:right;" | ||
|- | |- | ||
| − | |0|| | + | |0||6||11||04 |
|} | |} | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{ | + | ::<span style=color:Green>Rule of Three:</span> If 40 dinar earn 11, how much will 6 earn? |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{40:11=6:a_1}}</math> |
| − | + | |style="text-align:right;"|אם המ' דינר הרויחו י"א הו' כמה ירויחו | |
| − | |||
|- | |- | ||
| − | |||
| | | | ||
| + | ::We multiply the means; it is 66. | ||
| + | |style="text-align:right;"|נכפול האמצעיים ונהיו ס"ו | ||
|- | |- | ||
| | | | ||
| − | : | + | ::We divide it by 40, which is the extreme; the quotient is one, a half, an eighth, and one part of forty; and so the owner of the 6 dinar takes. |
| − | : | + | ::<math>\scriptstyle{\color{blue}{a_1=\frac{11\sdot6}{40}=\frac{66}{40}=1+\frac{1}{2}+\frac{1}{8}+\frac{1}{40}}}</math> |
| − | :<math>\scriptstyle\frac{1}{2} | + | |style="text-align:right;"|ונחלקם על מ' שהוא הקצה ונהיה החלק הא' אחד וחצי ושמיני' וחלק אחד מארבעים וככה יקח בעל הו' דינרים |
| − | |style="text-align:right;"| | ||
| − | |||
|- | |- | ||
| | | | ||
| − | :: | + | ::We write also the ratio of the second as follows: |
| − | |style=" | + | |style="text-align:right;"|עוד נכתוב הערך של השני ככה |
|- | |- | ||
| | | | ||
| − | :: | + | ::{|class="wikitable" style="text-align:right; color:blue" |
| − | |style="text-align:right;"| | + | |- |
| + | |0||7||11||40 | ||
| + | |} | ||
| + | |style="text-align:right;"| | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:right;" | ||
|- | |- | ||
| − | | | + | |0||7||11||04 |
| − | + | |} | |
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | :: | + | ::<span style=color:Green>Rule of Three:</span> If 40 dinar earn 11, how much will 7 earn? |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{40:11=7:a_2}}</math> |
| + | |style="text-align:right;"|אם המ' דינר הרויחו י"א הז' דינרים כמה ירויחו | ||
|- | |- | ||
| | | | ||
| − | ::We | + | ::We multiply the means; it is 77. |
| − | + | |style="text-align:right;"|נכפל האמצעיים ונהיו ע"ז | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | : | + | ::We divide it by the extreme, which is 40; the quotient is one, 3-quarters, an eighth, and one part of twenty; and so the owner of the 7 dinar takes. |
| − | ::<math>\scriptstyle{\color{blue}{\ | + | ::<math>\scriptstyle{\color{blue}{a_2=\frac{11\sdot7}{40}=\frac{77}{40}=1+\frac{3}{4}+\frac{1}{8}+\frac{1}{20}}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|נחלקם על הקצה שהוא המ' ונהיה החלק הא' אחד וג' רביעיות ושמינית וחלק אחד מעשרים וככה יקח בעל הז' דינרים |
|- | |- | ||
| | | | ||
| − | :: | + | ::We write also the ratio of the third as follows: |
| − | + | |style="text-align:right;"|עוד נכתוב הערך של השלישי ככה | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | | | + | ::{|class="wikitable" style="text-align:right; color:blue" |
| + | |- | ||
| + | |0||8||11||40 | ||
| + | |} | ||
| + | |style="text-align:right;"| | ||
{|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:right;" | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:right;" | ||
|- | |- | ||
| − | |0|| | + | |0||8||11||04 |
|} | |} | ||
|- | |- | ||
| | | | ||
| − | :{{ | + | ::<span style=color:Green>Rule of Three:</span> If 40 dinar earn 11, how much will 8 earn? |
| − | + | ::<math>\scriptstyle{\color{blue}{40:11=8:a_3}}</math> | |
| − | :<math>\scriptstyle\frac{ | + | |style="text-align:right;"|אם המ' דינרי' הרויחו י"א הח' דינרים כמה ירויחו |
| − | |style="text-align:right;"| | + | |- |
| + | | | ||
| + | ::We multiply the means; it is 88. | ||
| + | |style="text-align:right;"|נכפל האמצעיים ונהיו פ"ח | ||
| + | |- | ||
| + | | | ||
| + | ::We divide it by 40, which is the extreme; the quotient is 2 and a fifth; and so the owner of the 8 dinar takes. | ||
| + | ::<math>\scriptstyle{\color{blue}{a_3=\frac{11\sdot8}{40}=\frac{88}{40}=2+\frac{1}{5}}}</math> | ||
| + | |style="text-align:right;"|נחלקם על המ' שהוא הקצה ונהיה החלק האחד ב' וחמישית וככה יקח בעל הח' דינר | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::We write also the ratio of the fourth as follows: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|עוד נכתוב ערך הרביעי כך |
|- | |- | ||
| | | | ||
| − | :: | + | ::{|class="wikitable" style="text-align:right; color:blue" |
| − | |style="text-align:right;"| | + | |- |
| + | |0||9||11||40 | ||
| + | |} | ||
| + | |style="text-align:right;"| | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:right;" | ||
| + | |- | ||
| + | |0||9||11||04 | ||
| + | |} | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::<span style=color:Green>Rule of Three:</span> If 40 dinar earn 11, how much will 9 earn? |
| − | ::<math>\scriptstyle{\color{blue}{ | + | ::<math>\scriptstyle{\color{blue}{40:11=9:a_4}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|‫<ref>40r</ref>אם המ' דינר הרויחו י"א הט' כמה ירויחו |
|- | |- | ||
| | | | ||
| − | ::We multiply the means | + | ::We multiply the means; it is 99. |
| − | |style="text-align:right;"|נכפול האמצעיים | + | |style="text-align:right;"|נכפול האמצעיים ונהיו צ"ט |
|- | |- | ||
| | | | ||
| − | ::We divide it by | + | ::We divide it by 40; the quotient is 2, a quarter, a fifth, and one part of forty; and so the owner of the 9 dinar takes. |
| − | ::<math>\scriptstyle{\color{blue}{ | + | ::<math>\scriptstyle{\color{blue}{a_4=\frac{11\sdot9}{40}=\frac{99}{40}=2+\frac{1}{4}+\frac{1}{5}+\frac{1}{40}}}</math> |
| − | |style="text-align:right;"|נחלקם על | + | |style="text-align:right;"|נחלקם על המ' שהוא הקצה ונהיה החלק הא' ב' ורביעית וחמישית וחלק א' מארבעי' וככה יקח בעל הט' דינר |
|- | |- | ||
| | | | ||
| − | :: | + | ::We write also the ratio of the fifth as follows: |
| − | + | |style="text-align:right;"|עוד נכתוב הערך של החמישי ככה | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
::{|class="wikitable" style="text-align:right; color:blue" | ::{|class="wikitable" style="text-align:right; color:blue" | ||
|- | |- | ||
| − | |0|| | + | |0||10||11||40 |
|} | |} | ||
|style="text-align:right;"| | |style="text-align:right;"| | ||
{|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:right;" | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:right;" | ||
|- | |- | ||
| − | |0|| | + | |0||01||11||04 |
|} | |} | ||
| − | |||
| − | |||
|- | |- | ||
| − | |||
| | | | ||
| + | ::<span style=color:Green>Rule of Three:</span> If 40 dinar earn 11, how much will 10 earn? | ||
| + | ::<math>\scriptstyle{\color{blue}{40:11=10:a_5}}</math> | ||
| + | |style="text-align:right;"|אם המ' דינרים הרויחו י"א הי' כמה ירויחו | ||
|- | |- | ||
| − | | | + | | |
| − | : | + | ::We multiply the means; it is 110. |
| − | + | |style="text-align:right;"|נכפול האמצעיים ונהיו ק"י | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |style="text-align:right;"| | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | :: | + | ::We divide it by 40, which is the extreme; the quotient is 2 and three-quarters; and so the owner of the 10 dinar takes. |
| − | ::<math>\scriptstyle{\color{blue}{40 | + | ::<math>\scriptstyle{\color{blue}{a_5=\frac{11\sdot10}{40}=\frac{110}{40}=2+\frac{3}{4}}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|נחלקם על מ' שהוא הקצה ונהיה החלק האחד ב' ושלש רביעיות וככה יקח בעל הי' דינרים |
| − | |||
|- | |- | ||
| | | | ||
| − | ::the | + | :*<span style=color:Green>Check:</span> When you sum all the shares that they take, you find them 11, no more and no less. |
| − | ::<math>\scriptstyle{\color{blue}{ | + | |style="text-align:right;"|וכשתקבץ כל החלקים שיקחו הכל תמצאם י"א לא פחות ולא יתר |
| − | + | |- | |
| + | |colspan=2| | ||
| + | ::<math>\scriptstyle{\color{blue}{\begin{align}&\scriptstyle a_1+a_2+a_3+a_4+a_5\\&\scriptstyle=\left(1+\frac{1}{2}+\frac{1}{8}+\frac{1}{40}\right)+\left(1+\frac{3}{4}+\frac{1}{8}+\frac{1}{20}\right)+\left(2+\frac{1}{5}\right)+\left(2+\frac{1}{4}+\frac{1}{5}+\frac{1}{40}\right)+\left(2+\frac{3}{4}\right)=11\\\end{align}}}</math> | ||
| + | |||
|- | |- | ||
| | | | ||
| − | :: | + | :*Another method to find this: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<big>דרך</big> אחרת למצוא זה |
|- | |- | ||
| − | | | + | | |
| + | ::Convert the 11 dinar that are the total profit into pešuṭim, then divide them by 40 and you will see how much is the profit of each dinar of the 40. | ||
| + | |style="text-align:right;"|תעשה הי"א דינר שהם הריוח כלם פשוטים ותחלקם על מ' ותראה כמה ריוח יגיע לכל דינר מהמ‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | ::*Multiply this profit by 6 and the result is the share of the owner of the 6 dinar. |
| − | + | |style="text-align:right;"|והריוח ההוא תכפול אותו עם הו' ואשר יהיה ככה יגיע לבעל הו‫' | |
| − | |||
| − | |||
| − | |||
| − | |style="text-align:right;"|'' | ||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::*Multiply it by 7 and the result is the share of the owner of the 7 dinar. |
| − | + | |style="text-align:right;"|עוד תכפול אותו עם הז' ואשר יהיה ככה יגיע לבעל הז‫' | |
|- | |- | ||
| − | | | + | | |
| − | + | ::*Do likewise with 8, 9, and 10. | |
| + | |style="text-align:right;"|וכן תעשה עם הח' והט' והי‫' | ||
|- | |- | ||
| + | !<span style=color:Green>Purchase Problem - Equal Amount</span> | ||
| | | | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :{{#annot:three coins|642|oglX}}10) Question: the buyer had three [kinds of] coins. |
| − | |style="text-align:right;"| | + | :He went to the seller to buy one liṭra of silk. |
| + | :Of the first coin it was worth 3 dinar. | ||
| + | :Of the second coin it was worth 4 dinar. | ||
| + | :Of the third coin it was worth 6 dinar. | ||
| + | :The buyer said: give me one liṭra of silk and take from my three coins equally so that you will take the value of the liṭra | ||
| + | :How much should he take from each coin? | ||
| + | |style="text-align:right;"|<big>י</big> <big>שאלה</big> יש אצל הקונה ג' מטבעים<br> | ||
| + | והלך אצל המוכר [לקנות]‫<ref>P1031 om.</ref> ליטרא אחת משי<br> | ||
| + | ומהמטבע הא' היתה שוה ג' דינרים<br> | ||
| + | ומהמטבע השני היתה שוה ד' דינרים<br> | ||
| + | ומהמטבע השלישי היתה שוה ו' דינרים<br> | ||
| + | ואמר הקונה תתן לי הליטרא משי וקח משלש מטבעותי בשווי עד שתקח מה ששוה הליטרא<br> | ||
| + | כמה יקח מכל מטבע ומטבע{{#annotend:oglX}} | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{ | + | ::<span style=color:Green>common denominator:</span> We look for the denominator; it is 72, because the product of 3 by 4 is 12 and the product of 12 by 6 is 72. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{3\sdot4\sdot6=12\sdot6=72}}</math> |
| + | |style="text-align:right;"|נבקש המורה כי הוא ע"ב שהרי כפל ג' על ד' י"ב וכפל י"ב על ו' ע"ב | ||
|- | |- | ||
| | | | ||
| + | ::Its third is 24; its quarter is 18; its sixth is 12; the total sum is fifty-four dinar. | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(\frac{1}{3}\sdot72\right)+\left(\frac{1}{4}\sdot72\right)+\left(\frac{1}{6}\sdot72\right)=24+18+12=54}}</math> | ||
| + | |style="text-align:right;"|ושלישיתו כ"ד ורביעיתו י"ח וששיתו י"ב והכל חמשים וארבע וזהו הדינר | ||
| + | |- | ||
| | | | ||
| − | + | ::We divide the denominator, which is 72, by fifty-four; the quotient is one and a third; and so he will take from each coin. | |
| − | + | ::<math>\scriptstyle{\color{blue}{\frac{72}{54}=1+\frac{1}{3}}}</math> | |
| − | + | |style="text-align:right;"|נחלק המורה שהוא ע"ב על חמשים וארבע ונהיה החלק הא' א' [ושליש]‫<ref>P1031 ורביע</ref> וככה יקח מכל מטבע | |
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | :: | + | :*We convert the dinar into 12 perutot and he takes 16 perutot from each [type of] coin: |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{12\sdot\left(1+\frac{1}{3}\right)=16}}</math> |
| − | + | |style="text-align:right;"|והנה נעשה הדינר י"ב פרוטות ויקח י"ו פרוטות מכל מטבע | |
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | :: | + | ::If you want to know how: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<big>ואם</big> תרצה לדעת איך הוא כך |
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{ | + | ::*Think as if he [wants to] take 36 perutot from the coin of 3 dinar and he takes 16 perutot from it. |
| − | |style="text-align:right;"| | + | :::<math>\scriptstyle{\color{blue}{12\sdot3=36}}</math> |
| + | |style="text-align:right;"|תחשוב כאלו לקח ל"ו [פרוטות]‫<ref>P1031 דינרים</ref> ממטבע ששוה ג' דינרים ולקח ממנו י"ו פרוטו‫' | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{ | + | ::*When he takes 16 perutot from the coin of 4 dinar, it is as if he takes 12 perutot from the coin of 3 dinar, as the ratio of 4 to 3. |
| − | |style="text-align:right;"| | + | :::<math>\scriptstyle{\color{blue}{4:3=16:12}}</math> |
| + | |style="text-align:right;"|וכשלקח עוד י"ו פרוטות ממטבע ששוה ד' דינרים כאלו לקח י"ב פרוטות ממטבע ששוה ג' דינרים כערך הד' אל הג‫' | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{ | + | ::We sum up 12 with 16; it is 28. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{16+12=28}}</math> |
| + | |style="text-align:right;"|נחבר הי"ב עם הי"ו נהיו כ"ח | ||
|- | |- | ||
| | | | ||
| − | :* | + | ::*When he takes 16 perutot from the coin of 6 dinar, it is as if he took 8 perutot from the coin of 3 dinar, as the ratio of 3 to 6. |
| − | |style="text-align:right;"| | + | :::<math>\scriptstyle{\color{blue}{6:3=16:8}}</math> |
| + | |style="text-align:right;"|עוד כשלקח י"ו פרוטות ממטבע ששוה ו' דינרים הוא כאלו לקח ח' פרוטות ממטבע ששוה ג' דינרים כערך הג' אל הו‫' | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{ | + | ::We sum up 8 with 28; it is 36 and it is the required. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{28+8=36}}</math> |
| + | |style="text-align:right;"|נחבר הח' עם הכ"ח ונהיו ל"ו וזהו המבוקש | ||
|- | |- | ||
| + | !<span style=color:Green>Payment Problems</span> | ||
| | | | ||
| − | |||
| − | |||
|- | |- | ||
| + | !<span style=color:Green>constructing a shelf</span> | ||
| | | | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | :{{#annot: | + | :{{#annot:constructing a shelf|612|nNEd}}11) Question: a man agreed with a craftsman to construct for him a shelf of 6 in length and 6 in width and he will pay him 8 dinar. |
| − | + | :He constructed for him [a shelf of] 3 in length and 3 in width. | |
| − | : | + | :How much should he pay him? |
| − | + | |style="text-align:right;"|<big>יא</big> <big>שאלה</big> אדם התנה עם אומן לבנות לו אצטבא ו' באורך ו' ברחב ויתן לו ח' דינרים<br> | |
| − | : | + | והוא בנה לו ג' באורך ג' ברחב<br> |
| − | + | כמה יתן לו{{#annotend:nNEd}} | |
| − | |||
| − | |style="text-align:right;"| | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :He will be paid a quarter |
| − | + | :<math>\scriptstyle{\color{blue}{{\color{red}{\frac{8\sdot3\sdot3}{6\sdot6}=8\sdot}}\frac{1}{2}\sdot\frac{1}{2}={\color{red}{8\sdot}}\frac{1}{4}}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אין ספק כי יתן לו הרביעי' שהוא ב' דינרים כי כפל חצי [על חצי]‫<ref>P1031 om.</ref> הוא רביעית אחד |
|- | |- | ||
| | | | ||
| − | : | + | :If he had constructed a half of the length by the whole width or half of the width by the whole length, he would have been paid a half of the payment |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|שאלו היה בונה כל האורך עם חצי הרחב או ההפך היה נותן לו המחצית |
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\ | + | *{{#annot:constructing a shelf|612|wMvn}}If he agreed with him to construct for him [a shelf of] 5 in length, 6 in width, and 7 in height and he will pay him 8 dinar. |
| − | |style="text-align:right;"| | + | :He constructed for him [a shelf of] 4 in length, 5 in width, and 6 in height. |
| + | :How much should he pay him? | ||
| + | :<math>\scriptstyle\frac{5\sdot6\sdot7}{8}=\frac{4\sdot5\sdot6}{X}</math> | ||
| + | |style="text-align:right;"|אבל אם התנה עמו שיבנה לו ה' באורך ו' ברחב ז' בגובה ויתן לו ח' דינרים<br> | ||
| + | והוא בנה ד' באורך [וה' ברחב]‫<ref>P1031 om.</ref> ו' בגובה<br> | ||
| + | כמה יתן לו{{#annotend:wMvn}} | ||
| + | |- | ||
| + | | | ||
| + | ::We should sum up all the numbers of the condition, then turn them into ratios, and likewise the measures of the construction: | ||
| + | |style="text-align:right;"|אז ראוי שנחבר כל מספרי התנאי ונשיבם אל הערכים וכן מספרי הבנין | ||
| + | |- | ||
| + | | | ||
| + | ::We sum up first the numbers of the condition; the sum is 18. | ||
| + | ::<math>\scriptstyle{\color{blue}{5+6+7=18}}</math> | ||
| + | |style="text-align:right;"|חברנו בתחלה מספרי התנאי ונהיו י"ח | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::We sum up also the measures of the construction; the sum is 15. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{4+5+6=15}}</math> |
| + | |style="text-align:right;"|עוד חברנו מספרי הבנין ונהיו ט"ו | ||
|- | |- | ||
| | | | ||
| − | ::<span style=color:Green>Rule of | + | ::<span style=color:Green>Rule of Three:</span> We take the ratio as follows: If 18 yield 8 dinar, how much will 15 yield? |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{18:8=15:X}}</math> |
| + | |style="text-align:right;"|ועשינו הערך ככה אם הי"ח יתנו ח' דינרי' הט"ו כמה יתנו | ||
|- | |- | ||
| | | | ||
::{|class="wikitable" style="text-align:right; color:blue" | ::{|class="wikitable" style="text-align:right; color:blue" | ||
|- | |- | ||
| − | |0|| | + | |0||15||8||18 |
|} | |} | ||
|style="text-align:right;"| | |style="text-align:right;"| | ||
{|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:right;" | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:right;" | ||
|- | |- | ||
| − | |0|| | + | |0||51||8||81 |
|} | |} | ||
|- | |- | ||
| | | | ||
| − | ::We multiply the means; it is | + | ::We multiply the means; it is 120. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כפלנו האמצעיים ונהיו ק"כ |
|- | |- | ||
| | | | ||
| − | ::We divide it by | + | ::We divide it by 18, which is the extreme; the quotient is 6 and 2-thirds; and so he is paid. |
| − | ::<math>\scriptstyle{\color{blue}{ | + | ::<math>\scriptstyle{\color{blue}{X=\frac{8\sdot15}{18}=\frac{120}{18}=6+\frac{2}{3}}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|חלקנום על הי"ח שהוא הקצה ונהיה החלק ו' וב' שלישיות וככה יקח |
|- | |- | ||
| + | !<span style=color:Green>three workers, three different daily wages, the same actual payment</span> | ||
| | | | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | ::{| | + | :{{#annot:three workers|612|XpkI}}12) Question: a man hired three brothers - Reuven, Shimon and Levi - for two days so that one of them will work with him. |
| + | :If Reuven will work with him the whole two days he will pay him 6 dinar. | ||
| + | :If Shimon will work alone he will pay him 4 dinar. | ||
| + | :If Levi will work alone he will pay him 3 dinar. | ||
| + | :They worked a total of two days together. | ||
| + | :Finally, he paid each of them an equal share. | ||
| + | :How much is the share of each of them and how many hours did each of them work? | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle 6a_1=4a_2=3a_3\\\scriptstyle a_1+a_2+a_3=2\end{cases}</math> | ||
| + | |style="text-align:right;"|<big>יב</big> <big>שאלה</big> אדם א' שכר שלשה אחים ראובן שמעון לוי לעבוד אחד מהם עמו ב' ימים<br> | ||
| + | ואם יעבוד ראובן עמו כל הב' ימים יתן לו ו' דינרי‫'<br> | ||
| + | ואם יעבוד שמעון לבדו יתן לו ד' דינרי‫'<br> | ||
| + | ואם יעבוד לוי לבדו יתן לו ג' דינרי‫'<br> | ||
| + | עתה בין הכל עבדו הב' ימים<br> | ||
| + | ובאחרונה יפרע לכל חלק שוה<br> | ||
| + | כמה לקח כל אחד ואחד וכמה שעות עבד כל אחד{{#annotend:XpkI}} | ||
|- | |- | ||
| − | | | + | | |
| − | + | ::<span style=color:Green>common denominator:</span> We look for the denominator; it is 72, because the product of 6 by 4 is 24, and this by 3 is 72. | |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{6\sdot4\sdot3=24\sdot3=72}}</math> |
| − | + | |style="text-align:right;"|נבקש המורה והוא ע"ב כי כפל ו' על ד' הם כ"ד ואלה על ג' הם ע"ב | |
|- | |- | ||
| − | | | + | | |
| − | + | ::Its sixth, its third, and its quarter are 54. | |
| + | ::<math>\scriptstyle{\color{blue}{\left(\frac{1}{6}\sdot72\right)+\left(\frac{1}{3}\sdot72\right)+\left(\frac{1}{4}\sdot72\right)=54}}</math> | ||
| + | |style="text-align:right;"|וששיתו ורביעיתו ושלישיתו הם נ"ד | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::As the ratio of 72 to 54, so one is paid from the dinar. The ratio of 72 to 54 is one and a third; and so each of them is paid. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{72:54=1+\frac{1}{3}}}</math> |
| + | |style="text-align:right;"|‫[הנה כערך ע"ב אל נ"ד]‫<ref>P1031 om.</ref> ככה יקח ‫<ref>40v</ref>מהדינרים וערך ע"ב אל נ"ד הוא אחד ושליש וככה יקח כל אחד ואחד | ||
|- | |- | ||
| | | | ||
| − | ::We | + | ::We wish to know how many hours each of them worked: |
| − | : | + | |style="text-align:right;"|נבקש לדעת כמה שעות עבד כל [אחד ואחד]‫<ref>P1031 om.</ref> |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | :: | + | :::We turn it into ratios and convert the dinar into thirds, because of the third that is added to the dinar. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ונשיבהו אל הערכים ונשיב הדינר שלישיות מפני השליש שעודף על הדינרים |
|- | |- | ||
| | | | ||
| − | :: | + | :::The day is 12 hours. |
| − | + | |style="text-align:right;"|והיום י"ב שעות | |
| − | |||
| − | |||
| − | |style="text-align:right;"| | ||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | ::We | + | ::<span style=color:Green>Rule of Three:</span> We take Reuven's ratio as follows: If 18-thirds are 24 hours, how much will 4-thirds yield? |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\frac{18}{3}:24=\frac{4}{3}:a_1}}</math> |
| + | |style="text-align:right;"|ונעשה ערך ראובן כך אם הי"ח שלישיות כ"ד שעות הד' שלישיות כמה יתנו | ||
|- | |- | ||
| | | | ||
| − | ::We | + | ::We multiply the means; it is 96. |
| − | + | |style="text-align:right;"|ואז נכפול האמצעיים ונהיו צ"ו | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::We divide it by 18; the quotient is 5 hours and a third; and so Reuven worked. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{a_1=\frac{24\sdot4}{18}=\frac{96}{18}=5+\frac{1}{3}}}</math> |
| + | |style="text-align:right;"|נחלקם על י"ח ונהיה החלק ה' שעות ושליש וככה עבד ראובן | ||
|- | |- | ||
| | | | ||
::{|class="wikitable" style="text-align:right; color:blue" | ::{|class="wikitable" style="text-align:right; color:blue" | ||
|- | |- | ||
| − | |0|| | + | |0||4||24||18 |
|} | |} | ||
|style="text-align:right;"| | |style="text-align:right;"| | ||
{|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:right;" | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:right;" | ||
|- | |- | ||
| − | |0|| | + | |0||4||42||81 |
|} | |} | ||
|- | |- | ||
| | | | ||
| − | ::We | + | ::<span style=color:Green>Rule of Three:</span> We take Shimon's ratio as follows: If 12-thirds are 24 hours, how much will 4-thirds yield? |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\frac{12}{3}:24=\frac{4}{3}:a_2}}</math> |
| + | |style="text-align:right;"|ונעשה ערך שמעון כך אם הי"ב שלישיות כ"ד שעות הד' שלישיות כמה יתנו | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::{|class="wikitable" style="text-align:right; color:blue" |
| − | : | + | |- |
| − | + | |0||4||24||12 | |
| + | |} | ||
| + | |style="text-align:right;"| | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:right;" | ||
| + | |- | ||
| + | |0||4||42||21 | ||
| + | |} | ||
|- | |- | ||
| | | | ||
| − | : | + | ::We multiply the means; it is 96. |
| − | + | |style="text-align:right;"|כפלנו האמצעיים והם צ"ו | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::We divide it by 12; the quotient is 8 hours and so Shimon worked. |
| + | ::<math>\scriptstyle{\color{blue}{a_2=\frac{24\sdot4}{12}=\frac{96}{12}=8}}</math> | ||
| + | |style="text-align:right;"|חלקנום על י"ב ונהיה החלק ח' שעות וככה עבד שמעון | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::<span style=color:Green>Rule of Three:</span> We take Levi's ratio as follows: If 9-thirds yield 24 hours, how much will 4-thirds yield? |
| + | ::<math>\scriptstyle{\color{blue}{\frac{9}{3}:24=\frac{4}{3}:a_3}}</math> | ||
| + | |style="text-align:right;"|ונעשה ערך לוי כך אם הט' שלישיות יתנו כ"ד שעות הד' שלישיות כמה יתנו | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;" | + | ::{|class="wikitable" style="text-align:right; color:blue" |
|- | |- | ||
| + | |0||4||24||9 | ||
|} | |} | ||
| − | {| | + | |style="text-align:right;"| |
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:right;" | ||
|- | |- | ||
| − | | | + | |0||4||42||9 |
| − | + | |} | |
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | = | + | ::We multiply the means; it is 96. |
| − | + | |style="text-align:right;"|כפלנו האמצעים ונהיו צ"ו | |
| − | | | ||
|- | |- | ||
| | | | ||
| − | + | ::We divide it by 9; the quotient is 10 hours and 2-thirds; and so Levi worked. | |
| − | + | ::<math>\scriptstyle{\color{blue}{a_3=\frac{24\sdot4}{9}=\frac{96}{9}=10+\frac{2}{3}}}</math> | |
| − | + | |style="text-align:right;"|חלקנום על ט' ונהיה החלק י' שעות וב' שלישיים וככה עבד לוי | |
| − | |||
| − | |||
| − | |style=" | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*<span style=color:Green>Check:</span> Sum up all the hours that the three of them worked and you will find that they are 24 hours, which are two days. |
| + | ::<math>\scriptstyle{\color{blue}{a_1+a_2+a_3=\left(5+\frac{1}{3}\right)+8+\left(10+\frac{2}{3}\right)=24}}</math> | ||
| + | |style="text-align:right;"|חבר כל השעות שעבדו שלשתם ותמצאם כ"ד שעות שהם שני ימים | ||
|- | |- | ||
| + | !<span style=color:Green>Boiling Problem</span> | ||
| | | | ||
| − | |||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | :{{#annot:WP|663|7Xxv}}13) Question: a man was boiling 6 measures of a beverage and he wanted that one third will remain. One measure was evaporated while cooking, then one measure overflow and four measures remained. |
| + | :He wants to [reduce] them [as planned for the original amount] | ||
| + | :<math>\scriptstyle\frac{6-1}{\frac{1}{3}\sdot6}=\frac{4}{X}</math> | ||
| + | |style="text-align:right;"|<big>יג</big> שאלה אדם אחד היה מבשל משקה ו' מדות ורצה שישאר השליש ונחסרה המדה האחת בבשול אחר נשפכה מדה אחת ונשארו הד' מדות<br> | ||
| + | ורוצה להשאירם כמשפט הראשון{{#annotend:7Xxv}} | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | ::<span style=color:Green>Rule of Three:</span> We turn them into ratios as follows: if 5 gives 2, how much will 4 give? |
| + | ::<math>\scriptstyle{\color{blue}{5:2=4:X}}</math> | ||
| + | |style="text-align:right;"|נשיבם אל הערכים ככה אם הה' יתנו ב' הד' כמה יתנו | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:right;" | ||
|- | |- | ||
| − | | | + | |0||4||2||5 |
| − | | | + | |} |
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | ::We multiply the means; it is 8. We divide it by the extreme, which is 5; the quotient is 1, a half, and a tenth; and so should remain. |
| + | ::<math>\scriptstyle{\color{blue}{X=\frac{2\sdot4}{5}=\frac{8}{5}=1+\frac{1}{2}+\frac{1}{10}}}</math> | ||
| + | |style="text-align:right;"|נכפול האמצעיים ונהיו ח' נחלקם על הקצה שהוא הה' ונהיה החלק א' וחצי ועשירית וככה ראוי שישאר | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | :14) Question: a sheet of 100 cubits long. |
| + | :Reuven began to measure it from one end and Shimon from the other end. | ||
| + | :Reuven measures 10 cubit in one hour and Shimon [measures] 12 [cubit in one hour] | ||
| + | :When will they meet? | ||
| + | |style="text-align:right;"|<big>יד</big> שאלה יריעה מאה אמות<br> | ||
| + | והתחיל למנותה ראובן מן הקצה האחד ושמעון מן הקצה הא‫'<br> | ||
| + | וראובן מונה י' אמות בשעה ושמעון י"ב<br> | ||
| + | מתי יפגשו | ||
|- | |- | ||
| − | | | + | | |
| − | + | ::We sum up the two counts that are 22 cubits in one hour. We divide the 100 cubits by it; the quotient is 4, a half, and one part of 22. So, we know that in 4 hours, a half, and one part of 22 of a hour the two will meet in their count. | |
| − | + | ::<math>\scriptstyle{\color{blue}{\frac{100}{10+12}=\frac{100}{22}=4+\frac{1}{2}+\frac{1}{22}}}</math> hours | |
| − | + | |style="text-align:right;"|נחבר שני המנינים אשר הם כ"ב אמות בשעה ונחלק הק' אמות עליהם ונהיה החלק ד' וחצי וחלק א' מכ"ב<br> | |
| − | + | וידענו שבד' שעות וחצי וחלק מן כ"ב בשעה יפגשו שניהם על המנין | |
| − | |||
| − | |||
| − | |style="text-align:right;"| | ||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::If you want to know how many cubits Reuven counts: |
| + | |style="text-align:right;"|<big>ואם</big> תרצה לדעת כמה אמות מנה ראובן | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Multiply 10 by 4, a half, and one part of 22; the result is the number of cubits that Reuven count. |
| + | ::<math>\scriptstyle{\color{blue}{10\sdot\left(4+\frac{1}{2}+\frac{1}{22}\right)}}</math> | ||
| + | |style="text-align:right;"|תכפול י' בד' וחצי וחלק אחד מכ"ב ואשר יעלה כן מספר האמות אשר מנה ראובן | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::If you want to know how many cubits Shimon counts: |
| + | |style="text-align:right;"|וכן אם תרצה לדעת כמה מנה שמעון | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|ואשר | + | ::Multiply 4, a half, and one part of 22 by 12; the result is the number of cubits that Shimon count. |
| + | ::<math>\scriptstyle{\color{blue}{12\sdot\left(4+\frac{1}{2}+\frac{1}{22}\right)}}</math> | ||
| + | |style="text-align:right;"|תכפול הד' שעות וחצי וחלק מכ"ב על י"ב ואשר יעלה כך מספר האמות אשר מנה שמעון | ||
|- | |- | ||
| + | !<span style=color:Green>Shared Work Problem - Draining a Vessel - Barrel</span> | ||
| | | | ||
| − | |||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | :15) Question: a full barrel has three taps |
| + | :If the first is opened the barrel is drained in half a day. | ||
| + | :If the second is opened it is drained in a third of a day. | ||
| + | :If the third is opened it is drained in a quarter of a day. | ||
| + | :If you opened all three taps together, in how many hours will the barrel to be drained? | ||
| + | :<math>\scriptstyle\frac{1}{\frac{1}{2}}x+\frac{1}{\frac{1}{3}}x+\frac{1}{\frac{1}{4}}x=1</math> | ||
| + | |style="text-align:right;"|<big>טו</big> <big>שאלה</big> חבית מלאה יש בה שלשה נקבים<br> | ||
| + | אם תפתח האחד יכלה החבית בחצי היום<br> | ||
| + | ואם תפתח השנית יכלה בשלישית היום<br> | ||
| + | ואם תפתח השלישי יכלה ברביעית היום<br> | ||
| + | פתחת השלשה נקבים ביחד בכמה שעות יכלה החבית | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::You should know that the day is 12 hours. |
| + | |style="text-align:right;"|אתה צריך לדעת כי היום י"ב שעות | ||
|- | |- | ||
| | | | ||
| − | + | ::Also, how many measures does the barrel contain: we assume that it contains 48 measures. | |
| − | + | |style="text-align:right;"|וכן החבית כמה מדות מכילה ונניח שמכילה מ"ח מדות | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |style="text-align:right;"| | ||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::By opening the first tap it is drained in 6 hours [<math>\scriptstyle{\color{blue}{\frac{1}{2}\sdot12=6}}</math>] that are 8 measures [<math>\scriptstyle{\color{blue}{\frac{48}{6}=8}}</math>] in one hour. |
| + | |style="text-align:right;"|והנה בפתיחת הנקב הא' יעלה בו' שעות שהם ח' מדות בשעה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::By opening the second tap it is drained in 4 hours [<math>\scriptstyle{\color{blue}{\frac{1}{3}\sdot12=4}}</math>] that are 12 measures [<math>\scriptstyle{\color{blue}{\frac{48}{4}=12}}</math>] in one hour. |
| + | |style="text-align:right;"|ובפתיחת הנקב השני יכלה בד' שעות שהם י"ב מדות בשעה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::By opening the third tap it is drained in 3 hours [<math>\scriptstyle{\color{blue}{\frac{1}{4}\sdot12=3}}</math>] that are 16 measures [<math>\scriptstyle{\color{blue}{\frac{48}{3}=16}}</math>] in one hour. |
| + | |style="text-align:right;"|ובפתיחת הנקב השלישי יכלה בג' שעות שהם י"ו מדות בשעה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Sum up all the measures that are drained in one hour; they are 36. |
| + | ::<math>\scriptstyle{\color{blue}{8+12+16=36}}</math> | ||
| + | |style="text-align:right;"|תחבר כל המדות אשר יכלו בשעה אחת והם ל"ו | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Divide 48 by 36; it is one and a [third]; so in one hour and a [third] all is drained [through all three taps]. |
| + | ::<math>\scriptstyle{\color{blue}{x=\frac{48}{36}=1+\frac{1}{3}}}</math> | ||
| + | |style="text-align:right;"|תחלק המ"ח על הל"ו יהיה אחד ורביע [נ"א ושליש]‫<ref>P1031 marg.</ref> והנה בשעה א' ורביע [ושליש]‫<ref>P1031 marg.</ref> יכלה הכל | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::<span style=color:Green>Rule of Three:</span> We apply the ratios as follows - if 36 is one hour, how much is 48? |
| + | ::<math>\scriptstyle{\color{blue}{36:1=48:x}}</math> | ||
| + | |style="text-align:right;"|ונעשה הערכים ככה אם הל"ו שעה אחת המ"ח על כמה יהיה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;" | + | ::{|class="wikitable" style="text-align:right; color:blue" |
|- | |- | ||
| − | | | + | |0||48||1||36 |
| − | |style="text-align:right;"| | + | |} |
| + | |style="text-align:right;"| | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:right;" | ||
|- | |- | ||
| − | | | + | |0||84||1||63 |
| − | | | + | |} |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::We multiply the means; it is 48. We divide it by the extreme; the quotient is one and a third. |
| + | ::<math>\scriptstyle{\color{blue}{x=\frac{48\sdot1}{36}=1+\frac{1}{3}}}</math> | ||
| + | |style="text-align:right;"|נכפול האמצעיים והם מ"ח נחלקם על הקצה שהם ל"ו ונהיה החלק הא' אחד ושלישית | ||
|- | |- | ||
| + | !<span style=color:Green>Find a Quantity Problems - Whole from Parts Problems</span> | ||
| | | | ||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :{{#annot:lance|650|6Rrb}}16) Question: a lance, a half of it is in the water, a third of it is in the soil, and up above [the water] it is 6 cubits long. |
| + | :What is the length of the lance? | ||
| + | :<math>\scriptstyle\frac{1}{2}X+\frac{1}{3}X+6=X</math> | ||
| + | |style="text-align:right;"|טז <big>שאלה</big> רומח חציו במים שלישיתו בעפר ולמעלה ו' אמות<br> | ||
| + | כמה גבהות כל הרומח{{#annotend:6Rrb}} | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::<span style=color:Green>False Position - common denominator:</span> We look for the denominator; it is 6. |
| + | |style="width:45%; text-align:right;"|נבקש המורה והוא ו‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Its half and its third are 5. |
| + | |style="text-align:right;"|וחציו ושלישיתו ה‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::We subtract them from the 6; 1 remains. |
| + | ::<math>\scriptstyle{\color{blue}{6-\left(\frac{1}{2}\sdot6\right)-\left(\frac{1}{3}\sdot6\right)=6-5=1}}</math> | ||
| + | |style="text-align:right;"|חסרם מן הו' ונשארו א‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::We multiply the 6 by the 6 that are the means. |
| + | |style="text-align:right;"|נכפול הו' עם הו' שהם האמצעיים | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::We divide it by the 1 that is the extreme; it is 36 and so is the length of the lance. |
| + | ::<math>\scriptstyle{\color{blue}{x=\frac{6\sdot6}{1}=36}}</math> | ||
| + | |style="text-align:right;"|ונחלקם על הא' שהוא הקצה נהיו ל"ו וככה גובה הרומח | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | :*<span style=color:Green>Check:</span> Its half is 18; its third is 12; it is 30 and up above it is 6. |
| + | ::<math>\scriptstyle{\color{blue}{\left(\frac{1}{2}\sdot36\right)+\left(\frac{1}{3}\sdot36\right)+6=18+12+6=30+6=36}}</math> | ||
| + | |style="text-align:right;"|וחציו י"ח ושלישיתו י"ב הרי ל' ולמעלה ו‫' | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | ::<span style=color:Green>Rule of Three:</span> We do the relation as follows: if 1 gives 6, how much will the 6 give? |
| + | ::<math>\scriptstyle{\color{blue}{1:6=6:x}}</math> | ||
| + | |style="text-align:right;"|ונעשה הערך כך אם הא' יתנו ו' הו' כמה יתנו | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;" | + | | |
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:right;" | ||
|- | |- | ||
| − | | | + | |0||6||6||1 |
| − | | | + | |} |
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | :{{#annot:tree|650|tHbk}}17) Question: a tree, a fifth of it is [ingrained] in the soil, a sixth of it is in the water, and up above the water it is 7 cubits long. |
| + | :What is the height of the tree? | ||
| + | :<math>\scriptstyle\frac{1}{5}X+\frac{1}{6}X+7=X</math> | ||
| + | |style="text-align:right;"|יז <big>שאלה</big> אילן חמישיתו בעפר וששיתו במים ולמעלה מהמים ז' אמות כמה גבהות כל האילן{{#annotend:tHbk}} | ||
|- | |- | ||
| − | |The | + | | |
| − | |style="text-align:right;"| | + | ::<span style=color:Green>False Position - common denominator:</span> The denominator is 30. |
| + | |style="text-align:right;"|‫<ref>41r</ref>הנה המורה ל‫' | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | ::Its fifth and its sixth are 11. |
| + | |style="text-align:right;"|וחמישיתו וששיתו י"א | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | ::We subtract them from 30, which is the denominator; 19 remains. |
| + | ::<math>\scriptstyle{\color{blue}{30-\left(\frac{1}{5}\sdot30\right)-\left(\frac{1}{6}\sdot30\right)=30-11=19}}</math> | ||
| + | |style="text-align:right;"|נגרעם מל' שהוא המורה נשארו י"ט | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | ::We multiply the means, which is 7 by 30; it is 210. |
| + | |style="text-align:right;"|נכפול האמצעיים שהם הז' בל' נהיו ר"י | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | ::We divide it by 19; it is 11 and one part of 19, and so is the height of the tree. |
| + | ::<math>\scriptstyle{\color{blue}{x=\frac{7\sdot30}{19}=\frac{210}{19}=11+\frac{1}{19}}}</math> | ||
| + | |style="text-align:right;"|נחלקם על י"ט ונהיו י"א חלקים וחלק א' מי"ט וככה גבהות האילן | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | ::<span style=color:Green>Rule of Three:</span> We do the relation as follows: |
| + | ::<math>\scriptstyle{\color{blue}{19:30=7:x}}</math> | ||
| + | |style="text-align:right;"|ונעשה ערך כך | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;" | + | ::{|class="wikitable" style="text-align:right; color:blue" |
|- | |- | ||
| − | | | + | |0||7||30||19 |
| − | |style="text-align:right;"| | + | |} |
| + | |style="text-align:right;"| | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:right;" | ||
|- | |- | ||
| − | | | + | |0||7||03||91 |
| − | | | + | |} |
|- | |- | ||
| − | + | !<span style=color:Green>Triangulation Problem - Two towers</span> | |
| − | + | | | |
|- | |- | ||
| − | |The | + | | |
| − | |style="text-align:right;"| | + | :{{#annot:two birds|655|kLvl}}18) Question: there are two towers, the height of one is 60 cubits and the height of the other is 40 cubits. |
| + | :The distance between them is 50 cubits. | ||
| + | :At the top of each tower sits a bird. | ||
| + | :Between them a water pool. | ||
| + | :The birds are flying at the same speed, going down and drinking at the same time, then taking off at the same time. | ||
| + | :How far is the pool from the base of each tower? | ||
| + | :<math>\scriptstyle40^2+\left(50-a\right)^2=60^2+a^2</math> | ||
| + | |style="text-align:right;"|'''יח''' <big>שאלה</big> אם היו שני מגדלים גובה האחד ששים אמות וגובה השני מ' אמות<br> | ||
| + | והמרחק שביניהם נ' אמה<br> | ||
| + | ובראש כל מגדל צפור יושב<br> | ||
| + | ובין המגדלים בריכת מים<br> | ||
| + | ועפים הצפרים בהתעופפות שוה ויורדים ושותים בזמן שוה ועולים בזמן שוה<br> | ||
| + | כמה מרחק הבריכה מיסוד כל מגדל{{#annotend:kLvl}} | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::<span style=color:Green>denominator:</span> We add the square of 40, which is 1600, to the square of 50, which is 2500; it is 4100. We take the square of 60, which is 3600, and subtract it from the two squares mentioned that are 4100; 500 remains and this is the denominator. |
| + | |style="text-align:right;"|נחבר מרובע מ' שהוא אלף ת"ר עם מרובע נ' שהוא אלפים ות"ק נהיו ד' אלפים ק‫'<br> | ||
| + | אחר כן נקח מרובע ס' שהוא ג' אלפים ות"ר ונגרעם מן שני המרובעים הנזכרים שהם ד' אלפים ק' ונשארו ת"ק והוא המורה | ||
|- | |- | ||
| − | | | + | | colspan="2"| |
| − | + | ::<math>\scriptstyle{\color{blue}{40^2+50^2-60^2=1600+2500-3600=4100-3600=500}}</math> | |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::We multiply the distance between the two towers, which is 50, by 2; it is 100. We divide the denominator by it; it is 5 and this is the distance between the pool and the tower whose height is 60 cubits. |
| + | ::<math>\scriptstyle{\color{blue}{a=\frac{40^2+50^2-60^2}{2\sdot50}=\frac{500}{100}=5}}</math> | ||
| + | |style="text-align:right;"|נכפול גם מרחק שני המגדלי' שהוא נ' על ב' ונהיו ק' נחלק המורה עליהם ונהיו ה' וככה מרחק הבריכה מן המגדל שגבהו ס' אמות | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::[The distance between the pool and] the other tower is the remaining 45 cubits. |
| + | ::<math>\scriptstyle{\color{blue}{50-a=50-5=45}}</math> | ||
| + | |style="text-align:right;"|ומן המגדל האחר המ"ה אמות הנשארות | ||
|- | |- | ||
| − | + | !<span style=color:Green>Joint Purchase Problem - If You Give Me - Buying a Fish</span> | |
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :{{#annot:fish|664|6wf6}}19) Question: three men went to the market to buy a fish. |
| + | :The first said: I will give all of what I have and you will give a half of what you have and we will buy the fish. | ||
| + | :The second said: I will give all of what I have and you will give a third of what you have and we will buy the fish. | ||
| + | :The third said: I will give all of what I have and you will give a quarter of what you have and we will buy the fish. | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle a_1+\frac{1}{2}\sdot\left(a_2+a_3\right)=N\\\scriptstyle a_2+\frac{1}{3}\sdot\left(a_1+a_3\right)=N\\\scriptstyle a_3+\frac{1}{4}\sdot\left(a_1+a_2\right)=N\end{cases}</math> | ||
| + | |style="text-align:right;"|<big>‫[יט] שאלה</big> שלשה אנשים אשר הלכו בשוק לקנות דג<br> | ||
| + | אמר הא' אני אתן כל מה שיש לי ואתם תנו החצי ממה שיש לכם ונקח הדג<br> | ||
| + | אמר השני אני אתן כל אשר לי ואתם תנו השליש ממה שיש לכם ונקח הדג<br> | ||
| + | ‫[אמר הג' אני אתן כל מה שיש ואתם תנו הרביע ממה שיש לכם ונקנה הדג]‫<ref>P1031 om.</ref>{{#annotend:6wf6}} | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Write the words of the buyers: the half, the third, and the quarter, as follows: |
| + | |style="text-align:right;"|כתוב דבר הקונים החצי והשליש והרביע כזה | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:right;" | ||
|- | |- | ||
| − | | | + | |4||3||2 |
| − | | | + | |} |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Then, say: what is [the number] that when we take its half from it, 3 remains, as the number of buyers? It is 6. Write it beneath the 2. |
| + | ::<math>\scriptstyle{\color{blue}{a-\left(\frac{1}{2}\sdot a\right)=3\longrightarrow a=6}}</math> | ||
| + | |style="text-align:right;"|אחר כן תאמר מהו כאשר נקח חציו ממנו ישאר ג' כמספר הקונים והנה הוא הו' כתוב אותו תחת הב‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Say also: what is [the number] that when you take its third from it, 3 remains, as the number of buyers? It is 4 and a half. Write it beneath the 3. |
| + | ::<math>\scriptstyle{\color{blue}{b-\left(\frac{1}{3}\sdot b\right)=3\longrightarrow b=4+\frac{1}{2}}}</math> | ||
| + | |style="text-align:right;"|עוד תאמר מהו כאשר תקח שלישיתו ממנו ישאר ג' כמספר הקונים והנה הוא ד' וחצי כתוב אותו תחת הג‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Say also: what is [the number] that when you take its quarter from it, 3 remains, as the number of buyers? It is 4. Write it beneath the 4. |
| + | ::<math>\scriptstyle{\color{blue}{c-\left(\frac{1}{4}\sdot c\right)=3\longrightarrow c=4}}</math> | ||
| + | |style="text-align:right;"|עוד תאמר מהו כאשר תקח רביעיתו ממנו ישאר ג' כמספר הקונים והנה הוא ד' תכתבהו תחת הד‫' | ||
|- | |- | ||
| | | | ||
| − | |||
| − | |||
| | | | ||
| − | |style="text-align:right;" | + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:right;" |
|- | |- | ||
| − | | | + | |4||4||6 |
| − | | | ||
|- | |- | ||
| − | | | + | | ||<math>\scriptstyle\frac{1}{2}</math>|| |
| − | + | |} | |
| − | |||
| − | |||
| − | | | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::<span style=color:Green>common denominator:</span> Multiply all by 2 and say: 2 times 6 is 12; 2 times 4 and a half is 9; 2 times 4 is 8. Sum up all; it is 29 and this is the denominator. |
| + | ::<math>\scriptstyle{\color{blue}{\left(2\sdot6\right)+\left[2\sdot\left(4+\frac{1}{2}\right)\right]+\left(2\sdot4\right)=12+9+8=29}}</math> | ||
| + | |style="text-align:right;"|כפול הכל עם הב' ותאמר ב' פעמים ו' הם י"ב<br> | ||
| + | עוד ב' פעמים ד' וחצי הם ט‫'<br> | ||
| + | עוד ב' פעמים ד' הם ח‫'<br> | ||
| + | חבר הכל הם כ"ט וזהו המורה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Multiply the first, which is 12, by 2 and subtract it from the denominator; 5 remains and this is what the first has in his purse. |
| + | ::<math>\scriptstyle{\color{blue}{a_1=29-\left(2\sdot12\right)=5}}</math> | ||
| + | |style="text-align:right;"|כפול הראשון שהוא י"ב עם ב' וחסרהו מן המורה נשארו ה' וכך יש לראשון בכיסו | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Multiply the second, which is 9, by 2 and subtract it from the denominator; 11 remains and this is what the second has in his purse. |
| + | ::<math>\scriptstyle{\color{blue}{a_2=29-\left(2\sdot9\right)=11}}</math> | ||
| + | |style="text-align:right;"|כפול השני שהוא ט' עם הב' וחסרהו מן המורה נשארו י"א וכך יש לשני בכיסו | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Multiply the third, which is 8, by 2 and subtract it from the denominator; 13 remains and this is what the third has in his purse. |
| + | ::<math>\scriptstyle{\color{blue}{a_3=29-\left(2\sdot8\right)=13}}</math> | ||
| + | |style="text-align:right;"|כפול השלישי שהוא ח' עם ב' וחסרהו מן המורה ונשארו י"ג וכך יש לשלישי בכיסו | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::We find that they bought the fish for 17. |
| + | ::<math>\scriptstyle{\color{blue}{N=17}}</math> | ||
| + | |style="text-align:right;"|נמצא שקנו הדג בי"ז | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*Check: If you want to check it: |
| + | |style="text-align:right;"|ואם תרצה לבחון זה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::See that the first gave all that he has, which is 5, and they gave half of what they have. What they have is 24, because the second has 11 and the third 13; its half is 12. Add it to the 5; it is 17 and this is the price of the fish. |
| + | |style="text-align:right;"|תביט כי הראשון נתן כל מה שיש לו והיו ה' והם נתנו החצי שיש להם ומה שיש להם הם כ"ד כי השני יש לו י"א והג' י"ג וחציים הוא י"ב תחברם עם הה' ונהיו י"ז [והוא מחיר הדג]‫<ref>P1031 om.</ref> | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | ::<math>\scriptstyle{\color{blue}{a_1+\frac{1}{2}\sdot\left(a_2+a_3\right)=5+\frac{1}{2}\sdot\left(11+13\right)=5+\left(\frac{1}{2}\sdot24\right)=5+12=17}}</math> | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Also, the second gave all that he has, which is 11, while the first and the third gave a third of what they have, which is 6. Because they both have 18: the first [has] 5 and the third 13. Add 11 to the 6; it is 17 and this is the price of the fish. |
| + | |style="text-align:right;"|עוד השני נתן כל אשר לו והם י"א והראשון והשלישי נתנו השליש ממה שיש להם שהם ו' כי לשניהם י"ח לראשון ה' ולשלישי י"ג חבר הי"א עם הו' ונהיו י"ז והם מחיר הדג | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | ::<math>\scriptstyle{\color{blue}{a_2+\frac{1}{3}\sdot\left(a_1+a_3\right)=11+\frac{1}{3}\sdot\left(5+13\right)=11+\left(\frac{1}{3}\sdot18\right)=11+6=17}}</math> | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|< | + | ::Also, the third gave all that he has, which is 13, while the first and the second gave 4, which is a quarter of what they have. Because the first [has] 5 and the second 11. So, the price of the fish is 17. |
| + | |style="text-align:right;"|עוד השלישי נתן כל אשר לו והם הי"ג והראשון עם השני נתנו ד' שהוא הרביע ממה שיש להם כי לראשון ה' ולשני י"א ומחיר הדג י"ז | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | ::<math>\scriptstyle{\color{blue}{a_3+\frac{1}{4}\sdot\left(a_1+a_2\right)=13+\frac{1}{4}\sdot\left(5+11\right)=13+\left(\frac{1}{4}\sdot16\right)=13+4=17}}</math> | ||
|- | |- | ||
| − | + | !<span style=color:Green>Divide a Quantity Problem - Proportional Division – Promissory Note</span> | |
| − | + | | | |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :{{#annot:promissory note|645|MBAv}}20) Question: four men issued a promissory note with a man. |
| + | :The first [was promised] that if he would work with him for a month, he will pay him 10 zehuvim. | ||
| + | :The second [was promised] that if he would work with him for a month, he will pay him 5 zehuvim which are a half. | ||
| + | :The third [was promised] that if he would work with him for a month, he will pay him 3⅓ zehuvim which are a third. | ||
| + | :The fourth [was promised] that if he would work with him for a month, he will pay him 2½ zehuvim which are a quarter. | ||
| + | :They all worked a whole month, but he did not pay any one and died. | ||
| + | :All that he had were only 10 zehuvim | ||
| + | |style="text-align:right;"|<big>‫[כ] שאלה</big> אדם אחד הוציאו עליו שטר חוב ארבעה אנשים<br> | ||
| + | הראשון אם יעבוד עמו חדש ימים יתן לו עשרה זהובים<br> | ||
| + | והשני אם יעבוד עמו חדש ימים יתן לו ה' זהובי' שהם מחציתם<br> | ||
| + | והשלישי שאם יעבוד עמו חדש ימים יתן לו ג' זהובים ושליש שהם שלישיתם<br> | ||
| + | והרביעי שאם יעבוד עמו חדש ימים יתן לו ב' זהובים וחצי שהם רביעיתם<br> | ||
| + | ועבדו כלם חדש א' בשלמות ולא פרע לשום א' ומת<br> | ||
| + | ולא נמצאו לו יותר מי' זהובים בכל אשר היה לו{{#annotend:MBAv}} | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::The arithmeticians give each according to his relative share. |
| + | ::<math>\scriptstyle X+\frac{1}{2}X+\frac{1}{3}X+\frac{1}{4}X=10</math> | ||
| + | |style="text-align:right;"|הנה חכמי החשבון יתנו לכל אחד כפי ערך ממונו | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::<span style=color:Green>False Position - common denominator:</span> The common denominator is twenty four, because if you multiply 2 by three; it is 6; then 6 by 4; it is 24 and in it all the mentioned parts are found. We define the whole unit as 24 because it is the denominator. |
| + | ::<math>\scriptstyle{\color{blue}{2\sdot3\sdot4=6\sdot4=24}}</math> | ||
| + | |style="text-align:right;"|והנה המורה עשרים וארבעה כי תכפול הב' על השלשה והם ו' עוד הו' על הד' הם כ"ד ובזה ימצאו כל החלקים הנזכרים ונשים האחד השלם כ"ד כי הוא המורה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::When you add to it its half, its third, and its quarter, the total is 50. |
| + | ::<math>\scriptstyle{\color{blue}{24+\left(\frac{1}{2}\sdot24\right)+\left(\frac{1}{3}\sdot24\right)+\left(\frac{1}{4}\sdot24\right)=50}}</math> | ||
| + | |style="text-align:right;"|וכשתחבר אליו חציו ושלישיתו ורביעיתו יהיה בין הכל נ‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::We convert the 10 zehuvim into 120 pešuṭim at 12 pešuṭim in one zahuv. <math>\scriptstyle{\color{blue}{12\sdot10=120}}</math> |
| + | |style="text-align:right;"|ונעשה הי' זהובים ק"כ פשוטים מי"ב פשוטים בזהוב | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|< | + | ::<span style=color:Green>Rule of three:</span> We do the ratio of the first as follows: if 50 gives [us] 24, how much will 120 give us? |
| + | ::<math>\scriptstyle{\color{blue}{50:24=120:a_1}}</math> | ||
| + | |style="text-align:right;"|ונעשה ערך הראשון ‫<ref>41v</ref>כך אם הנ' יתנו לו כ"ד הק"כ מה יתנו לנו | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;" | + | ::{|class="wikitable" style="text-align:right; color:blue" |
|- | |- | ||
| − | | | + | |0||120||24||50 |
| − | |style="text-align:right;"| | + | |} |
| + | |style="text-align:right;"| | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:right;" | ||
|- | |- | ||
| − | | | + | |0||021||42||05 |
| − | | | + | |} |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::We multiply the means; it is 2880. |
| + | |style="text-align:right;"|נכפול האמצעיים ונהיו ב' אלפים תת"פ | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::We divide it by 50, which is the extreme; the quotient is 57 pešuṭim, a half of a pašuṭ, and a tenth of a pašuṭ; this is the first share of the owner of the 10 zehuvim. |
| + | ::<math>\scriptstyle{\color{blue}{a_1=\frac{24\sdot120}{50}=\frac{2880}{50}=57+\frac{1}{2}+\frac{1}{10}}}</math> | ||
| + | |style="text-align:right;"|נחלקם על הנ' שהוא הקצה נהיה החלק נ"ז פשוטים וחצי פשוט ועשירי' פשוט וזהו החלק הראשון בעל הי' זהובים | ||
|- | |- | ||
| | | | ||
| − | + | ::<span style=color:Green>Rule of Three:</span> We do the ratio of the second as follows: if 50 gives us 12, how much will 120 give [us]? | |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{50:12=120:a_2}}</math> |
| + | |style="text-align:right;"|ונעשה הערך השני כך אם הנ' יתנו לנו י"ב הק"כ מה יתנו | ||
|- | |- | ||
| | | | ||
| − | : | + | ::{|class="wikitable" style="text-align:right; color:blue" |
| − | |style="text-align:right;" | ||
|- | |- | ||
| − | | | + | |0||120||12||50 |
| − | : | + | |} |
| − | |style="text-align:right;" | + | |style="text-align:right;"| |
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:right;" | ||
|- | |- | ||
| − | | | + | |0||021||21||05 |
| − | + | |} | |
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::We multiply the means; it is 1440. |
| + | |style="text-align:right;"|נכפול האמצעיים ונהיו אלף ת"מ | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::We divide it by 50, which is the extreme; the result is 28 pešuṭim, half a pašuṭ, a fifth of a pašuṭ, and a tenth of a pašuṭ; and this is the second share of the owner of the 5 zehuvim. |
| + | ::<math>\scriptstyle{\color{blue}{a_2=\frac{12\sdot120}{50}=\frac{1440}{50}=28+\frac{1}{2}+\frac{1}{5}+\frac{1}{10}}}</math> | ||
| + | |style="text-align:right;"|נחלקם על הנ' שהוא הקצה ועלו כ"ח פשוטי' וחצי פשוט וחמשית פשוט ועשירית פשוט וזהו חלק השני בעל הה' זהובים | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::<span style=color:Green>Rule of Three:</span> We do the ratio of the third as follows: if 50 yields 8, how much will 120 yield? |
| + | ::<math>\scriptstyle{\color{blue}{50:8=120:a_3}}</math> | ||
| + | |style="text-align:right;"|ונעשה ערך השלישי ככה אם הנ' יתנו ח' הק"כ כמה יתנו | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;" | + | ::{|class="wikitable" style="text-align:right; color:blue" |
|- | |- | ||
| − | | | + | |0||120||8||50 |
| − | |style="text-align:right;"| | + | |} |
| + | |style="text-align:right;"| | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:right;" | ||
|- | |- | ||
| − | | | + | |0||021||8||05 |
| − | | | + | |} |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::We multiply the means; it is 960. |
| + | |style="text-align:right;"|נכפול האמצעיים ונהיו תתק"ס | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::We divide it by 50, which is the extreme; it is 19 pešuṭim and a fifth, and this is the third share of the owner of the 3 zehuvim and a third. |
| + | ::<math>\scriptstyle{\color{blue}{a_3=\frac{8\sdot120}{50}=\frac{960}{50}=19+\frac{1}{5}}}</math> | ||
| + | |style="text-align:right;"|נחלקם על הנ' שהוא הקצה יהיו י"ט פשוטים וחמישית וזהו החלק השלישי בעל הג' זהובים ושליש | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::<span style=color:Green>Rule of Three:</span> We do the ratio of the fourth as follows: if 50 yields 6, how much will 120 yield? |
| + | ::<math>\scriptstyle{\color{blue}{50:6=120:a_4}}</math> | ||
| + | |style="text-align:right;"|ונעשה ערך הרביעי ככה אם הנ' יתנו ו' הק"כ כמה יתנו | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;" | + | ::{|class="wikitable" style="text-align:right; color:blue" |
|- | |- | ||
| − | | | + | |0||120||6||50 |
| − | |style="text-align:right;"| | + | |} |
| + | |style="text-align:right;"| | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:right;" | ||
|- | |- | ||
| − | | | + | |0||021||6||05 |
| − | | | + | |} |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::We multiply the means; it is 720. |
| + | |style="text-align:right;"|נכפול האמצעיים ונהיו תש"כ | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::We divide it by 50, which is the extreme; it is 14 pešuṭim and 2-fifths of a pašuṭ and this is the fourth share of the owner of the 2 zehuvim and a half. |
| + | ::<math>\scriptstyle{\color{blue}{a_4=\frac{6\sdot120}{50}=\frac{720}{50}=14+\frac{2}{5}}}</math> | ||
| + | |style="text-align:right;"|נחלקם על הנ' שהוא הקצה ונהיו י"ד פשוטים וב' חמישיות פשוט וזהו החלק הרביעי בעל הב' זהובים וחצי | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*<span style=color:Green>Check:</span> When you sum up all these shares, the result is 120 pešuṭim that are 10 zehuvim. |
| + | |style="text-align:right;"|וכשתחבר כל כל אלה החלקים יעלו ק"כ פשוטים שהם י' זהובים | ||
|- | |- | ||
| − | | | + | |colspan=2| |
| − | + | ::<math>\scriptstyle{\color{blue}{a_1+a_2+a_3+a_4=\left(57+\frac{1}{2}+\frac{1}{10}\right)+\left(28+\frac{1}{2}+\frac{1}{5}+\frac{1}{10}\right)+\left(19+\frac{1}{5}\right)+\left(14+\frac{2}{5}\right)=120}}</math> | |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :If you do not want to turn all into ratios because of the bother, do as follows: |
| + | |style="text-align:right;"|ואם לא תרצה להשיב הכל אל הערכים מפני הטורח תעשה כך | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :After you know the share of the first by the ratio, give its half to the second, its third to the third, and its quarter to the fourth; and it will be the same. |
| + | |style="text-align:right;"|אחר שידעת חלק הא' מן הערכים תן מחציתו לב' ושלישיתו לג' ורביעיתו לד' ושוה יהיה זה וזה | ||
|- | |- | ||
| − | | | + | |You can create other questions endlessly and you can answer any of them, if you know the rules we elaborated in this book. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכן תוכל אתה לעשות שאלות אחרות עד אין קץ ותוכל להשיב על כל אחת מהן אם תדע הכללים שהקפנו בהם בזה החבור |
|- | |- | ||
| − | | | + | |I have not mention a way to find the roots of the squares here, because they are not needed in this book, so I have postponed them to their place, where I will explain them, if the One ''Who gives strength to the weary'' [Isaiah 40, 29] wants. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ולא הזכרתי דרך מציאת שרשי המרובעים הנה כי אינם צורך בזה החבור ואחרתים עד מקומם ושם אבארם אם יחפוץ ''הנותן ליעף כח''‫<ref group=note>ישעיהו מ, כ"ט</ref>‫<ref>P1031 marg.: אנכי צעיר יחיאל<br>שאלני שואל מי שיש לו סך מה והוא יודע שהרויח כל כך במאה וע"י זה נתקבץ סך המעות ההם ועתה רוצה לדעת כמה היה הקרן והריוח כל אחד לבדו<br>התשובה בזה שתעריך כמה הוא הריוח מהמאה ותוסיף א' ותחלק כל המעות ויצא לך הריוח והשאר הוא הקרן<br>המשל היה סך המעות אלף ושמנה מאות ושבעים וחמש והריוח היה כ"ה במאה<br>נעריך כ"ה אצל המאה והוא רביע נקח ארבעה ועוד א' שהם חמשה נחלק כל הסך לחמשה ויצא שע"ה והוא הריוח והנשאר הוא הקרן<br> אלא שאם יהיה הריוח כ"ג או כ"ו שאז לא יש ערך שלם כי ערך הכ"ו אצל הק' הוא ג' וכ"ב מאיים שהם י"א חמישיים נוסיף א' יהיו ד' וי"א חמישיים אז נחלק המעות בדרך חלוק השברים על דרך זה המשל היה סך המעות</ref> |
| + | |- | ||
| + | |} | ||
| + | {| | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|<big> | + | |
| + | == The Second Book of this Treatise Discusses Geometry == | ||
| + | |||
| + | |style="width:45%; text-align:right;"|‫<ref>42r</ref><big>הספר השני מזה החבור המדבר בחכמת המדות</big> | ||
|- | |- | ||
| | | | ||
| − | + | === [The First Section] === | |
| − | + | ||
| − | + | | | |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|<big> | + | ==== Chapter One of the First Section of the Second Book ==== |
| + | |||
| + | |style="text-align:right;"|<big>הפרק הראשון</big> מהחלק הראשון מהספר השני | ||
|- | |- | ||
| − | | | + | |Mordechai said: before discussing geometry [lit. the science of measurement] it is appropriate to explain the names used by the masters of this craft and science, for in each science, the masters of this science use specific names that indicate issues of this science, not issues that are indicated in other than this science, as they are conventional. |
| − | |style="text-align:right;"| | + | |style="width:45%; text-align:right;"|<big>אמר מרדכי</big> קודם שנדבר על חכמת המדות ראוי לבאר שמות שנשתמשו בהם בעלי זאת המלאכה והחכמה כי לכל חכמה שמות מיוחדות נשתמשו בהם בעלי החכמה ההיא מורות בענינים באותה החכמה בלתי הענינים אשר יורו בזולת החכמה ההיא להיות שהם הסכמיים |
|- | |- | ||
| − | | | + | |As they are used in other than that science, they can be transferred to a specific science, either by metaphor or by analogy or by the translation, and sometimes even by complete similarity. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכאשר הניחו בזולת החכמה ההיא על מה שהניחו כן יוכלו להעתיקם ממה שהניחום למה שהניחום בחכמה מיוחדת אם על דרך השאלה או על דרך הספוק או על דרך ההעתקה ולפעמים גם ע"ד השתוף הגמור |
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |Therefore, if an author of a book wants his words to be understood, he should first explain the words used by the masters of this science. |
| − | + | |style="text-align:right;"|ולכן ראוי למחבר ספר אם ירצה להיות דבריו מובנים לבאר תחילה השמות אשר נשתמשו בם בעלי החכמה ההיא | |
| − | |||
| − | |||
| − | |style=" | ||
|- | |- | ||
| − | | | + | |So did the sage Euclid in the Book of Elements, who clarified in the introduction of each book the things that are necessary in that book. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכן עשה גם החכם אקלידס בספר היסודות אשר ביאר בהצעת כל מאמר דברים הצריכים באותו מאמר |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *We say that when you hear "a point" here, you understand that it indicates a thing that has no measure at all, no length, no breadth and no depth. It is found at the beginning of the line, because it is its limit, and it is what is found in the middle of the line when a notation estimates the limit of half the line and it is itself the beginning of the other half of the line. |
| + | |style="text-align:right;"|ונאמר שכשתשמע הנה נקדה תבין שתורה על דבר שאין לו שעור כלל לא ארך ולא רחב ולא עומק והיא נמצאת בראש הקו כי היא תכליתו וכן היא אשר תמצא באמצע הקו כשתשער רמיזה היא תכלית חצי הקו והיא בעצמה ראש חצי הקו האחר | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *The line is a length with no breadth drawn between two points that are its limits. |
| + | |style="text-align:right;"|והקו הוא אורך בלא רחב נמשך בין שתי נקדות אשר הן תכליותיו | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *The surface is a breadth stretched between lines, which has only length and breadth and its limits are the lines. |
| + | |style="text-align:right;"|והשטח הוא הרחב המתוח בין הקוים ויש לו אורך ורחב לבד ותכליותיו הם הקוים | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *The plane angle is that which is encompassed by two straight lines. |
| + | |style="text-align:right;"|והזוית השטוחה היא אשר יקיפו בה שני קוי' ישרי‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Among the angles there are right angles, acute angles and obtuse angles. |
| + | |style="text-align:right;"|והזוית ממנה נצבת וממה חדה וממנה נרוחת | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*The right angle is [generated] when a straight line falls on a straight line and makes the two angles on its either sides equal. |
| + | |style="text-align:right;"|והזוית הנצבת היא כשיפול הקו הישר על קו ישר וישים השתי זויות אשר משתי צדדיו שוות כזה ┴ | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*The acute angle is the one that is smaller than it. |
| + | |style="text-align:right;"|והחדה היא אשר היא יותר קטנה מזאת | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*The obtuse angle is the one that is greater than it. |
| + | |style="text-align:right;"|והנרוחת היא אשר היא יותר גדולה מזאת | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *When a line falls on a line and makes the two angles on its either sides equal, it is called perpendicular. |
| + | |style="text-align:right;"|והקו אשר יפול על הקו ויעשה שתי הזויות אשר משתי צדדיו שוות יקרא עמוד | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *The line on which it stands is called base. |
| + | |style="text-align:right;"|והקו שהוא עומד עליו יקרא תושבת | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *The parallel lines are those that extend to either side and do not meet even endlessly. |
| + | |style="text-align:right;"|והקוים הנכחיים הם אשר כשיוצאו לכל א' משני צדדים ואפילו ללא תכלית לא יפגשו | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *The plane shapes are divided into a polygon [lit. having straight lines] and non-polygonal [lit. not having straight lines]. |
| + | |style="text-align:right;"|והתמונה השטחית תחלק אל ישרת הקוים ואל בלתי ישרת הקוים | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*The polygon is that which is encompassed by three [straight] lines or more. |
| + | |style="text-align:right;"|והתמונה הישרת קוים היא אשר יקיפו בה ג' קוים או יותר | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::*That which is encompassed by three [straight] lines, no more, is called a triangle. |
| + | |style="text-align:right;"|ואשר יקיפו בה ג' קוים לא יותר תקרא משולש | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::*That which is encompassed by four [straight] lines is called a quadrangle. |
| + | |style="text-align:right;"|ואשר יקיפו בה ד' קוים תקרא מרובע | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::*That which is encompassed by five [straight] lines is called a pentagon. |
| + | |style="text-align:right;"|ואשר יקיפו בה ה' קוים תקרא מחומש | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::And so on and so forth |
| + | |style="text-align:right;"|וכן כל כיוצא בזה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *The lines encompassing the shape are called sides. |
| + | |style="text-align:right;"|והקוים אשר יקיפו בתמונה יקראו צלעות | ||
|- | |- | ||
| − | | | + | |The triangles are divided into three species: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והמשולש יחלק אל שלשה מינים |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *One is called equilateral, whose three sides are equal. |
| + | |style="text-align:right;"|הא' יקרא שוה הצלעות והוא אשר שלש צלעותיו שוים | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *The second is called isosceles, whose two sides are equal and the third is unequal, whether it is longer than both, or shorter than both. |
| + | |style="text-align:right;"|והשני יקרא שוה השוקים והוא אשר תהיינה שתי צלעותיו שוות והשלישית בלתי שוה אם יותר ארוכה מכל אחת מהן או יותר קצרה מכל אחת מהן | ||
|- | |- | ||
| − | |||
| | | | ||
| + | *The third is called scalene, which none [of its sides] is equal to the other. | ||
| + | |style="text-align:right;"|והשלישי יקרא מתחלף הצלעות והוא אשר אין אחת שוה לחברתה | ||
|- | |- | ||
| − | | | + | |The triangles are also divided into: |
| − | |style="text-align:right;"| | + | *Right triangle, whose one angle is a right angle, like this: |
| + | |style="text-align:right;"|עוד ‫<ref>42v</ref>המשולש יחלק אל נצב הזויות והוא אשר זויתו האחת נצבת כזה | ||
|- | |- | ||
| − | | | + | | |
| − | + | *Obtuse triangle, whose one angle is an obtuse angle, like this: | |
| − | + | |style="text-align:right;"|ואל נרוחת הזוית והוא שזויתו האחת מרווחת כזה | |
| − | |||
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | *Acute-angled triangle, whose three angles are acute angles. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואל חד הזויות והוא אשר שלש זויותיו חדות |
|- | |- | ||
| − | | | + | |The quadrangles are divided into: equilateral quadrangle and non-equilateral quadrangle. |
| − | + | |style="text-align:right;"|ובעל הד' צלעות יחלק אל מרובע שוה הצלעות ואל בלתי שוה הצלעות | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | :: | + | *The equilateral quadrangles are divided into: |
| − | |style="text-align:right;"| | + | :*Right-angled and it is called a square [lit. right-angled equilateral], like this: |
| + | |style="text-align:right;"|עוד השוה הצלעות יחלק אל מה שהוא נצב הזויות ויקרא שוה הצלעות נצב הזויות כזה | ||
|- | |- | ||
| | | | ||
| − | : | + | :*Not right-angled, like this, and it is called rhombus: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואל מה שהוא בלתי נצב הזויות כזה ויקרא מעויין |
|- | |- | ||
| | | | ||
| − | :: | + | *The non-equilateral quadrangles are divided into: |
| − | |style="text-align:right;"| | + | :*The one whose two parallel sides are equal and whose angles are right angles, which is called a rectangle [lit. long quadrangle], like this: |
| + | |style="text-align:right;"|עוד הבלתי שוה הצלעות יחלק אל מה שהוא שתי צלעותיו הנכוחיים שוים וזויותיו נצבות ויקרא מרובע ארוך כזה | ||
|- | |- | ||
| | | | ||
| − | : | + | :*The one whose two parallel sides are equal, but its angles are not right angles, which is called a parallelogram [lit. similar to a rhombus], like this: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואל [מה שכל שתי]‫<ref>P1031 כל ששתי</ref> צלעותיו [הנכוחות]‫<ref>P1031 om.</ref> שוות אבל אין זויותיו נצבות ויקרא דומה למעויין כזה |
|- | |- | ||
| | | | ||
| − | : | + | :*Other than these, which is called a trapezoid. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואל זולת אלה ויקרא הנוטה |
|- | |- | ||
| | | | ||
| − | :: | + | ::There are four [types of] shapes [of this category] that will be explained in this book. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והוא ארבע תמונות יתבארו בזה החבור |
|- | |- | ||
| | | | ||
| − | + | *The plane non-polygonal [lit. not having straight lines] shapes are divided into a circle and a semicircle. | |
| − | | | + | |style="text-align:right;"|והתמונה הבלתי ישרת הקוים השטוחה תחלק אל עגולה וחצי עגולה |
|- | |- | ||
| | | | ||
| − | : | + | :*The circle is a shape encompassed by one line and within it a point such that all the lines that are drawn from it to the perimeter are equal. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והעגולה היא תמונה יקיף בה קו אחד בתוכה נקדה כל הקוים היוצאים ממנה אל המקיף שוים |
|- | |- | ||
| | | | ||
| − | :: | + | ::*This point is called the center of the circle. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והנקדה ההיא תקרא מרכז העגולה |
|- | |- | ||
| | | | ||
| − | :: | + | ::*The line that is drawn from the perimeter of the circle, passes through the center and reaches the perimeter from the other side is called a diameter. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והקו [הישר]‫<ref>P1031 om.</ref> אשר יצא ממקיף העגולה ויעבור על המרכז [ויצא עד המקיף מהצד האחר יקרא הקוטר |
|- | |- | ||
| | | | ||
| − | :: | + | ::*If it does not pass through the center it is called chord. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואם לא יעבר על המרכז]‫<ref>P1031 om.</ref> יקרא מיתר |
|- | |- | ||
| | | | ||
| − | :: | + | ::*The segment whose base is a chord is called an arc. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והחתיכה אשר תושבתה במיתר יקרא קשת |
|- | |- | ||
| | | | ||
| − | : | + | :*The semicircle is encompassed by half the perimeter and the diameter. |
| − | | | + | |style="text-align:right;"|וחצי העגולה היא אשר יקיף בה חצי הקו המקיף והאלכסון |
|- | |- | ||
| | | | ||
| − | : | + | :*The segment of a circle is a shape encompassed by a chord and an arch and it is divided into two types: smaller than a semicircle or greater than it. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וחתיכת העגולה היא תמונה תקיף בה היתר והקשת ותחלק לשני מינים אם שהיא קטנה מחצי עגולה או גדולה הימנה |
|- | |- | ||
| | | | ||
| − | + | *The area of the shape is the right-angled quadrilateral parts, whose length is as their width, or those that are equal to them, that are filling the shape. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ותשבורת התמונה הוא החלקים המרובעים נצבי הזויות אשר ארכם כרחבם או השוים להם הממלאים את התמונה הדרושים על התמונה |
|- | |- | ||
| | | | ||
| − | : | + | *The multiplication is the product of a side by a side, or of a straight line by a curve, or of a number by a number. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וההכאה היא כפל צלע בצלע או קו ישר בקו קשתי או מספר במספר |
| + | |- | ||
| + | |Euclid has already explained in a book that he wrote about the measurement of land that the plane geometry is based on four things: directions, points, lines, and angles; and it also has species and types. | ||
| + | |style="text-align:right;"|וכבר ביאר אקלידס בספר אחד שחבר במדידת הארץ כי חכמת המדות השטוחה היא מיוסדת מארבעה דברים מהפאות ומהנקודות ומהקוים ומהזויות וגם כן היא מקבלת סוגים ומינים | ||
|- | |- | ||
| | | | ||
| − | + | *The directions are east, west, north, and south. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והפאות הם מזרח ומערב וצפון ודרום |
|- | |- | ||
| | | | ||
| − | + | *The points are those that you regard as a beginning or a sign of a thing. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והנקדות הם אשר תקח אותם התחלה או סימן לדבר |
|- | |- | ||
| | | | ||
| − | : | + | *The lines are ten: straight line, bottom base, upper base, perpendicular, parallel line, diagonal, legs, perimeter, diameter, and hypotenuse. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והקוים הם י' קו ישר ותושבת וראש ועמוד וקו נכחי וקטר ושוקים ומקיף ואלכסון ומיתר |
|- | |- | ||
| | | | ||
| − | : | + | :*The straight line is that which is drawn straightly from both its ends that are two points. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והקו הישר הוא הנמשך על יושר משתי תכליותיו שהם שתי נקדות |
|- | |- | ||
| | | | ||
| − | : | + | :*The bottom base is a straight line on which another straight line stands and makes the two angles on both its sides right angles |
| + | |style="text-align:right;"|והתושבת היא קו ישר אחד עומד עליו קו ישר אחר ועושה השתי זויות אשר משני צדדיו נצבות | ||
| + | |- | ||
| | | | ||
| + | :*The upper base is the line that stands above the base. | ||
| + | |style="text-align:right;"|והראש הוא הקו העומד על התושבת | ||
|- | |- | ||
| | | | ||
| − | : | + | :*The perpendicular is a line that goes down from the top to the base and makes the two angles on both its sides right angles. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והעמוד הוא הקו היורד מהראש אל התושבת ועושה השתי זויות אשר משתי צדדיו נצבות |
|- | |- | ||
| | | | ||
| − | : | + | :*The parallel line is a line that stands in parallel to another line and the distances of their extremes that are at right angles are equal. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והקו הנכחי הוא קו עומד נכח קו אחר ומרחקי קצותיהם אשר על זויות נצבות שוים |
|- | |- | ||
| | | | ||
| − | : | + | :*The diagonal is a line that is drawn from the one of the vertices of the quadrilaterals and their like to another vertex. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והקטר הוא הקו הנמשך מזויות המרובעים וכיוצא בהם עם הזוית האחרת |
|- | |- | ||
| | | | ||
| − | : | + | :*The legs are straight lines that descend from the top to both ends of the base. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והשוקים הם קוים ישרים יורדים מקצות [הראש]‫<ref>P1031 הארץ</ref> עד שתי קצות התושבת |
|- | |- | ||
| | | | ||
| − | : | + | :*The perimeter is the line that revolves at a fixed distance from the center to that line and the lines drawn to it from the center are equal. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והמקיף הוא הקו הסובב במרחק שהוא [מהמרכז]‫<ref>P1031 המרכז</ref> עד הקו ההוא והקוים היוצאים אליו מהמרכז שוים |
|- | |- | ||
| | | | ||
| − | : | + | :*The diameter is the line that is drawn from the perimeter [of the circle], passes through its center and reaches the perimeter from the other side. It divides the perimeter into two equal parts. |
| + | |style="text-align:right;"|והאלכסון הוא ‫<ref>43r</ref>קו יוצא מהמקיף ועובר על מרכזו ויוצא אל המקיף מהצד האחר וחולק את המקיף בשני חלקים שוים | ||
| + | |- | ||
| | | | ||
| + | :*The hypotenuse is a straight line that is opposite to the right angle. | ||
| + | |style="text-align:right;"|והמיתר הוא הקו הישר אשר תחת הזוית הנצבת | ||
|- | |- | ||
| − | |The | + | | |
| − | |style="text-align:right;"| | + | *The angles are three: right angle, acute angle and obtuse angle. |
| + | |style="text-align:right;"|והזויות הם ג' נצבת חדה ונרוחת | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | :*The right angle is such that when a straight line stands on a straight line the angles that are formed on both sides of it are equal, each being called right angle. |
| + | |style="text-align:right;"|הנצבת היא כאשר יעמוד קו ישר על קו ישר ויעשה הזויות אשר משני צדדיו שוות כל אחת תקרא נצבת | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | :*The angle that is smaller than this is called an acute angle. |
| + | |style="text-align:right;"|והזוית אשר היא קטנה מזאת תקרא חדה | ||
|- | |- | ||
| | | | ||
| − | :The | + | :*The one that is greater than this is called an obtuse angle. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואשר היא גדולה מזאת תקרא נרוחת |
|- | |- | ||
| | | | ||
| − | : | + | *The types of measurement are three: the measurement of straight lines, the measurement of the area of [plane] shapes, and the measurement of solids. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<big>סוגי</big> המדידה הם שלש [מדידות הקוים]‫<ref>P1031 מהארבעה קוים</ref> הישרי' ומדידת התמונה ר"ל תשבורת התמונות ומדידת הגופנים |
|- | |- | ||
| | | | ||
| − | : | + | :*The measurement of straight lines is the measurement by which they are measured straight according to length alone, and it is called a numerical measurement. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|מדידת הקוים הישרים היא [המדידה]‫<ref>P1031 om.</ref> אשר ימדדו על יושר באורך לבד וזה יקרא מדידה מספרית |
|- | |- | ||
| | | | ||
| − | : | + | :*The measurement of the area of [plane] shapes is the one that has length and breadth, from which the area of the shapes is known, and it is also called potential measurement. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|מדידת תשבורת התמונות [הוא אשר יש לו אורך ורחב כי ממנו יודעו תשברת התמונות ותקרא גם כן מדידה כחנית |
|- | |- | ||
| | | | ||
| − | : | + | :*The measurement of the solids is the one that has length, breadth, and thickness, from which the volume of the solids is known, and it is also called cube. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|מדידת הגופניים] הוא אשר יש לו אורך ורחב ועובי כי ממנו יודע מדידת הגופנים ויקרא גם כן מעוקב |
|- | |- | ||
| | | | ||
| − | :: | + | :*The types of area are five: quadrangle, triangle, rhombus, trapezoid, and circle. |
| − | + | |style="text-align:right;"|ומיני המדידה הם חמשה המרובע והמשולש והמעוין והנוטה והעגולות | |
| − | |||
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::The [plane] shapes are 18: |
| − | + | |style="text-align:right;"|והתמונות י"ח | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::*The shapes of the quadrangles are two: quadrangle whose sides are parallel, and right-angled quadrangle. |
| − | + | |style="text-align:right;"|אם תמונות המרובעים ב' מרובע נכחי הצלעות ונצב הזויות | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::*The shapes of the triangles are six: equilateral triangle, isosceles triangle, scalene triangle, right triangle, obtuse triangle, acute-angled triangle. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואם תמונות המשולשים [ששה]‫<ref>P1031 om.</ref> משולש שוה הצלעות ומשולש שוה השוקים ומשולש מתחלף הצלעות ומשולש נצב הזויות ומשולש נרוח הזויות ומשולש חד הזויות |
|- | |- | ||
| | | | ||
| − | :: | + | ::*The shapes of the rhombuses are two: rhombus, and parallelogram. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואם תמונות המעויינים ב' המעויין והדומה למעויין |
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | ::*The shapes of the trapezoids are four: right trapezoid, isosceles trapezoid, acute trapezoid, and obtuse trapezoid. |
| + | |style="text-align:right;"|ואם תמונות הנוטים הם ד' נוטה נצב הזויות נוטה שוה השוקים נוטה חד הזויות נוטה נרוח הזויות | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | ::*The shapes of the circles are four: circle, semicircle, segment of a circle that is greater than a semicircle, and segment of a circle that is smaller than a semicircle |
| + | |style="text-align:right;"|ואם תמונות העגולות ד' העגולה וחצי העגולה וחתיכת העגולה [הגדולה]‫<ref>P1031 om.</ref> מחציה ומעלה וחתיכת העגולה הקטנה שהיא קטנה ממנה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | |
| + | ==== Chapter Two <span style=color:Green>[on the Measuring of the Various Quadrangles]</span> ==== | ||
| + | |||
| + | |style="text-align:right;"|<big>הפרק השני</big> | ||
|- | |- | ||
| − | | | + | |Know that the triangle is the basis and foundation for all the planar shapes that consist of straight lines, for they are composed of it and decomposed to it, as Nicomachus of Gerasa stated in the second section of the Introduction to Arithmetic he wrote. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|דע כי המשולש הוא שרש ופנה לכל התמונות השטחיות ישרות הקוים כי ממנו חוברו ואליו יותכו כאשר הודיע זה {{#annot:Nicomachus|509|OiRc}}ניקומאכוש הגיהרשיני{{#annotend:OiRc}} במאמר השני מספר הארתימישיקא שחבר |
| + | |- | ||
| + | |Therefore, whoever knows how to measure the triangles knows how to measure all the shapes by multiplying the area of the triangle by the number that generates the change if they are regular and if they are irregular it is by summing the areas of all the triangles that generate them. | ||
| + | |style="text-align:right;"|ולכן מי שידע למדוד המשולשים ידע למדוד כל התמונות בכפל תשבורת המשולש במספר אשר יולידו התמורה אם הם שוים ואם היו בלתי שוים יהיה זה בקבוץ תשבורת כל המשולשי' אשר יולידוהו | ||
|- | |- | ||
| − | | | + | |Because of this it is enough to present the measuring of the triangle alone, however because the measure and the size by which we measure is a square, the length of which is equal to its width, for the area of the shapes is measured by the square cubits, since we know its definition, and the size of the triangle is verified only in comparison with the square, because its demonstration is obtained by comparison with the square. Hence, we should first introduce the measuring of the [squares], then we shall state the methods of the triangles and the others. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ומפני זה היה מספיק להודיע מדידת המשולש למנין לבד אבל מפני שהמדה והשעור אשר נשער בה מרובעת ארכה כרחבה כי תשבורת התמונות הם משוערות על האמות המרובעות כאשר הודענו מן גדרה והמשולש בעצמו לא יתאמת מנינו רק בהצטרפו אל המרובע כי המופת שיובא עליו בהקשה אל המרובע לכן ראוי שנודיע מדידת המשולשים [נ' המרובעים] תחלה אח"כ נודיע דרכי המשולשים והשאר |
|- | |- | ||
| − | | | + | |Before that, we announce that every unknown is obtained only through the known, therefore all those that are required are raised to the axioms or to one of the species which are known by themselves. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וקודם זה נודיע שכל מוסכל לא יגיע אלא בידוע ולכן עלו כל הדרושים אל המושכלות הראשונות או אל אחד מהמינים אשר הם ידועים בעצמם |
|- | |- | ||
| − | | | + | |It is known that when we seek to find the area of any shape, it is unknown to us before we find it. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וידוע כי כשנדרוש למצוא תשבורת תמונה איזו שתהיה קודם שנמצאנה היא מוסכלת אצלנו |
|- | |- | ||
| − | | | + | |Therefore, we should obtain it through the known and the known that we obtain is the knowledge of a part of it, whether one of its sides, its height, its base, or other than these. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ולכן ראוי להגיעה לנו בידוע והידוע אשר יגיע לנו היא ידיעת חלק ממנה אם צלעות מצלעותיה או עמוד ותושבת וזולת זה |
|- | |- | ||
| − | | | + | |If these are also unknown, then we obtain the cubits by which they are measured, so we should say that the known cubit, by which we obtain the measured that are unknown, is only length, without extension, it is not a square cubit, as the cubits of the area, for the ends of the square [cubit] are also lines that are only length and they limit it. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואם גם אלה הם מוסכלים אז תגיע לנו האמה אשר ימדדו בה ולכן ראוי להודיע ‫<ref>43v</ref>שהאמה הזאת ידועה אשר תגיע לנו ידיעת המוסכלים מהנמדדים היא אורך לבד בלי מרחב ואינה אמה מרובעת כאשר הם אמות התשבורת כי גם המרובעת תכליותיה הם הקוים אשר הם אורך לבד והם המגבילים אותה |
|- | |- | ||
| − | | | + | |From here I begin to explain how the quadrangles are measured and we start from the right-angled equilateral square, because it is as a foundation for the rest of the quadrangles, since the rhombus is similar to it by its equal sides and the rectangle by its right angles. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<big>ומהנה</big> אתחיל לבאר איך ימדדו המרובעים ונתחיל ממרובע שוה הצלעות נצב הזויות כי הוא כשרש לשאר המרובעים אחרי שהמעויין ידמה לו בשווי הצלעות והארוך בהתיצבות הזויות |
|- | |- | ||
| − | | | + | |The cubit of the area is square, equilateral and equiangular. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ועוד כי אמת התשבורת היא מרובעת הצלעות שוים והזויות |
|- | |- | ||
| − | | | + | |It is a cubit of the area, because it demonstrates for us the measure of the shape and the thing that demonstrates the measure of the thing should be equally known. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והיתה זאת אמת התשבורת כי זאת תודיע לנו השעור של התמונה והדבר אשר יודיע שעור הדבר ראוי שיהיה שוה נודע |
|- | |- | ||
| − | | | + | |It is known that the right angles are always equal, which is not the case for acute angle and obtuse angle that can be smaller or greater; and the thing that can be the smaller and greater is unlimited; and the unlimited is unknown; and the unknown itself cannot be a reason for other. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וידוע שהזויות הנצבות הם שוות לעולם מה שאין כן החדה והנרוחת כי יקבלו הפחות והיתר והדבר אשר יקבל הפחות והיתר הוא בלתי מוגבל והבלתי מוגבל הוא בלתי ידוע והבלתי ידוע בעצמו איך יהיה סבה לזולתו |
|- | |- | ||
| + | !<span style=color:Green>Finding the Area</span> | ||
| | | | ||
| − | |style="text-align:right;"| | + | |- |
| − | | | + | !The discussion on the measuring of the equilateral right-angled quadrangle [= square] |
| − | + | |style="text-align:right;"|<big>הדבור</big> על מדידת המרובע שוה הצלעות נצב הזויות | |
| + | |- | ||
| + | |You should know in general that for every right-angled quadrangle, its area is the product of its one side by its other side. | ||
| + | |style="text-align:right;"|ראוי שתדע בכלל כי כל בעל ארבעה צלעות נצב הזויות הארבעה הנה הכאת צלעו האחד בצלעו האחר ככה תשברתו | ||
| + | |- | ||
| + | |This is the reason that the square of its one side is equal to the product of its one side by its other side [sic.]. | ||
| + | |style="text-align:right;"|והיה זה סבה להיות שמרובע צלעו האחד שוה להכאת צלעו הא' בצלעו השני | ||
|- | |- | ||
| | | | ||
| − | + | *Example: an equilateral quadrangle [square] ABGD, we define each of its sides ten cubits. | |
| − | + | |style="text-align:right;"|דמיון מרובע אבג"ד הוא שוה הצלעות כי נשים כל א' מצלעותיו עשר אמות | |
| − | |||
| − | |style=" | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :The square of its one side is one hundred cubits and this is its area. |
| + | :<math>\scriptstyle{\color{blue}{10^2=100}}</math> | ||
| + | |style="text-align:right;"|והנה מרובע צלעו האחד מאה אמה וככה הוא תשברתו | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :For they are one hundred right-angled square cubits, each cubit of them is square and similar to the great square. |
| + | |style="text-align:right;"|כי הם מאה אמות מרובעות נצבות הזויות כל אמה מהן מרובעת דומה אל המרובע הגדול | ||
| + | |- | ||
| + | |Proof: we divide each of its side by ten cubits with ten points and we draw a line from each point on one side to the side parallel to it. | ||
| + | |style="text-align:right;"|<big>המופת</big> על זה נחלק כל צלע ממנו על עשר אמות בעשרה נקדות ונגיע מכל נקדה מהצלע האחד קו על הצלע הנכחי לו | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :These lines are parallel to each other and to the side of the large square, because the distances of their perpendicular ends are equal. |
| + | |style="text-align:right;"|הנה יהיו הקוים נכוחיים האחד לחברו ולצלעו המרובע הגדול כי מרחקי קצותיהם אשר הם על זויות נצבות שוים | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :So, <math>\scriptstyle HW\parallel AD</math>; <math>\scriptstyle KL\parallel AB</math> |
| + | |style="text-align:right;"|אם כן קו ה"ו נכחי אל קו א"ד וקו כ"ל נכחי אל קו א"ב | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :We say first that line AD falls on the two parallel lines AB and KL. |
| + | |style="text-align:right;"|ונאמר תחלה כי קו א"ד נפל על שני קוי א"ב וכ"ל הנכוחיים | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :The [sum of] two [consecutive] interior angles is equal to [the sum of] two right angles. |
| + | |style="text-align:right;"|הנה ישרת שתי הזויות הפנימיות אשר בצד אחד שוות לשתי נצבות | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :<math>\scriptstyle\measuredangle A=90^\circ</math> |
| + | |style="text-align:right;"|וזוית א' היא נצבת | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :So, <math>\scriptstyle\measuredangle K=90^\circ</math> |
| + | |style="text-align:right;"|אם כן גם זוית כ' היא נצבת על כל פנים | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :The opposite angles in parallelograms are equal. |
| + | |style="text-align:right;"|‫<ref>44r</ref>והתמונות הנכחיות הצלעות יהיו זויותיהם המומרות שוות | ||
|- | |- | ||
| − | |||
| | | | ||
| + | :So, <math>\scriptstyle\measuredangle H=90^\circ</math> | ||
| + | |style="text-align:right;"|א"כ גם זוית ה' נצבת | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | :The remaining angle of this small quadrangle, which is the cubit, is also right. |
| + | |style="text-align:right;"|וגם הזוית הנשארת מזה המרובע הקטן שהוא האמה נצבת | ||
|- | |- | ||
| | | | ||
| − | + | :Line HW falls on line AB. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|עוד קו ו"ה נפל על קו א"ב |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :When a straight line falls on a straight line, the two angles on its side [= supplementary angles] are either right angles, or [their sum is] equal to [the sum of] two right angles. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והקו הישר אשר יפול על קו ישר הנה ב' הזויות אשר בצדו אם נצבות או שוות לשתי נצבות |
|- | |- | ||
| | | | ||
| − | + | :<math>\scriptstyle\measuredangle H=90^\circ</math>, therefore the adjacent angle is also right angle. | |
| − | + | |style="text-align:right;"|וזוית ה' נצבת אם כן גם הזוית אשר בצדו נצבת | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | : | + | :It is clear from this that the four angles of the cubit are also right and so it is clear for all ten cubits of the first line. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ומזה יתבאר כי גם על ד' זויות האמות נצבות וכן יתבארו כל הי' אמות אשר בטור הא‫' |
|- | |- | ||
| | | | ||
| − | : | + | :Line KL is intersected by line line HW. |
| − | |style="text-align:right;"|עוד | + | |style="text-align:right;"|עוד קו כ"ל נחתך בקו ה"ו |
|- | |- | ||
| | | | ||
| − | : | + | :The vertical angles of two intersecting lines are equal. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וב' הקוים אשר יחתכו זויותיהם המקבילות שוות |
|- | |- | ||
| | | | ||
| − | : | + | :Therefore, the angle of the first cubit of the second line on the left is also right angle, and its opposite angle is right angle. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|א"כ גם זוית האמה הראשונה אשר בטור הב' השמאלית נצבת וכנגדה נצבת |
|- | |- | ||
| | | | ||
| − | : | + | :Similarly all the cubits of this area are verified. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכן יתבארו כל האמות אשר בתשבורת הזאת |
|- | |- | ||
| | | | ||
| − | : | + | :Their being equilateral is verified from the division of each side into equal parts, also because when you subtract [equal quantities] from equal [quantities], the remainders are equal and the two things that are equal to equal things are equal as well. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ולהיותם שוי הצלעות יתבאר מחלוקת כל צלע השוה לחלקים וכשתחסר מן השוים יהיה הנשאר שוים והב' דברים השוים לדברים שוים גם הם שוים |
|- | |- | ||
| + | !<span style=color:Green>Rectangle</span> | ||
| | | | ||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |This measuring is true for the right-angled quadrangle [= rectangle], i.e. that you multiply its one side by its other side that are encompassing one angle [= adjacent sides] and find the area. |
| − | + | |style="text-align:right;"|וזאת המדידה נכונה בבעלות ד' הנצבות הזויות הד' ר"ל שתכפול צלעו הא' על צלעו השני המקיפו' בזוית א' ולמצוא התשבורת | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | : | + | *{{#annot:rectangle|680|rG70}}Example of a right-angled quadrangle [= rectangle], one side of which is 10 and the other side is 5. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|דמיון בבעלת ד' צלעות נצב הזויות הד' נכוחי הצלעות אשר צלעה הא' י' וצלעה האחרת ה‫'{{#annotend:rG70}} |
|- | |- | ||
| | | | ||
| − | :: | + | :Like this: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כזה |
|- | |- | ||
| | | | ||
| − | : | + | :The product of 5 by 10 is 50 and this is the area of this shape. |
| − | + | :<math>\scriptstyle{\color{blue}{5\sdot10=50}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|הנה כפילת הה' על הי' הם נ' וזהו התשבורת של זאת התמונה |
|- | |- | ||
| | | | ||
| − | + | :Its proof is like the previous proof. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ומופתה כמופת הקודם |
|- | |- | ||
| + | !<span style=color:Green>Rhombus</span> | ||
| | | | ||
| − | + | |- | |
| − | |style="text-align:right;"| | + | |However, if the shape is equilateral but not right-angled, it cannot be measured by its sides, because it leads to a huge error. |
| + | |style="text-align:right;"|אבל אם היתה התמונה שוה הצלעות ולא היתה נצבת הזויות ולא יתכן שימדד במדידת צלעותיה כי זה יביא לטעות גדולה | ||
|- | |- | ||
| | | | ||
| − | : | + | *Example: we have an equilateral quadrangle ABGD that is not right-angled [= rhombus], each of its sides is 10 cubits. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|דמיון יש לנו מרובע שוה הצלעות ובלתי נצב הזויות עליו אבג"ד ויהיה כל א' מצלעיו י' אמות |
|- | |- | ||
| | | | ||
| − | : | + | :If you multiply its one side by its other side, or if you take the square of its one side, the result is 100 and this is not the area of this quadrangle. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|הנה אם תכפול צלעו הא' בצלעו השני אז אם תקח מרובע צלעו הא' יעלה ק' ואין זה תשבורת המרובע הזה |
|- | |- | ||
| − | | | + | |Proof: we draw two diagonals from its vertices: one is diagonal AG and the other is diagonal BD. |
| − | : | + | |style="text-align:right;"|<big>המופת</big> נוציא ב' קוטרים מזויותיו הא' קוטר א"ג והב' קטר ב"ד |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | : | + | :AG = 16 cubits; BD = 12 cubits. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ויהיה קטר א"ג י"ו אמה וקטר ב"ד י"ב אמה |
|- | |- | ||
| | | | ||
| − | : | + | :They intersect at point H. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ויחתכו על נקדת ה‫' |
|- | |- | ||
| | | | ||
| − | : | + | :A rectangle is formed, whose one side is as the long diagonal and its other side is as the short diagonal. |
| − | + | |style="text-align:right;"|הנה נעשה שטח נכוחי הצלעות נצב הזויות אשר צלעו אחת כקטר הארוך וצלעו האחרת כקטר הקצר | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | : | + | :Each side is parallel to its corresponding diagonal, because the two diagonals intersect at right angles as is demonstrated in its measure. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ויהיה כל צלע נכוחי לקטר הדומה לו כי שתי הקטרים יחתכו על זויות נצבות כאשר נראה המופת במדידתו |
|- | |- | ||
| | | | ||
| − | : | + | :We write on it: H, W, Z, C. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ונכתוב עליו ה' ו' ז' ח‫' |
|- | |- | ||
| | | | ||
| − | + | :So, the rectangle is divided into four equal parts by the diagonals of the small quadrangle whose sides are not right. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|הנה נחלק השטח הנצב הזויות לד' חלקים שוים עם קטרי המרובע הקטן אשר אין זויותיו נצבות |
|- | |- | ||
| | | | ||
| − | : | + | :Each of the four parts is also divided into two equal parts exactly by the sides of the small quadrangle. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|עוד כל חלק מהד' חלקים נחלק לשני חלקים שוים עם צלעות המרובע הקטן לא פחות ולא יתר |
|- | |- | ||
| | | | ||
| − | : | + | :The area of the rectangle is the product of its one side by the other. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ותשבורת השטח הנצב הזויות הוא בכפל צלעו האחד על השני |
|- | |- | ||
| | | | ||
| − | + | :Its one side is 16 cubits, because it is equal to the long diagonal of the quadrangle. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וצלעו הא' הוא י"ו אמה כי ‫<ref>44v</ref>הוא שוה לקטר הארוך מהמרובע |
|- | |- | ||
| | | | ||
| − | + | :Its other side is 12 cubits, because it is equal to the short diagonal of the quadrangle. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וצלעו השנית הוא י"ב אמה כי הוא שוה לקטר הקצר מהמרובע |
|- | |- | ||
| | | | ||
| − | : | + | :The product of 16 by 12 is 192 and this is the area of the rectangle, which is double the area of the quadrangle inside it. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{16\sdot12=192}}</math> |
| + | |style="text-align:right;"|וכפל י"ו על י"ב הם קצ"ב וזהו תשבורת השטח הנצב הזויות אשר הוא כפל תשבורת המרובע אשר בתוכו | ||
|- | |- | ||
| | | | ||
| − | : | + | :Hence, the area of the quadrangle inside it is 96 cubits. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואם כן תשבורת המרובע הם צ"ו אמות אשר בתוכו |
|- | |- | ||
| | | | ||
| − | : | + | :Had we relied on the product of its sides, the result would have been 100 cubits and this is a great mistake. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואלו היינו נשענים על כפל צלעותיו היה יוצא ק' אמה וזאת שגיאה גדולה |
|- | |- | ||
| − | | | + | |Therefore, the geometricians said that this quadrangle is measured by multiplying its one diagonal by the other diagonal and this is its area. |
| − | + | |style="text-align:right;"|ולכן אמרו בעלי השעור שזה המרובע יהיה נמדד בכפילת מחצית קטרו הא' על קטרו השני והוא תשברתו | |
| − | |||
| − | |style="text-align:right;"| | ||
|- | |- | ||
| − | | | + | |The proof of this measurement: it is known that since it is equilateral, its two diagonals intersect at right angles, because each diagonal divides it into two [equal] isosceles triangles and the diagonal is the base of the two triangles. |
| − | : | + | |style="text-align:right;"|<big>המופת</big> על המדידה הזאת ידוע שכאשר יהיה שוה הצלעות הנה שני קטריו יחתכו על זויות נצבות כי כל קטר הוא מחלק אותו לשני משולשים שוה השוקים והקטר תושבת שני המשולשים |
| − | |style="text-align:right;"| | + | |- |
| + | |When one diagonal is drawn from one vertex of the isosceles triangle opposite to the base to the other vertex of the [other] isosceles triangle opposite to the base, it divides the base also into two equal parts at right angles. | ||
| + | |style="text-align:right;"|וכאשר יצא הקטר האחד מזוית משולש שוה השוקים אשר התושבת היא מיתרה עד הזוית האחרת ממשולש שוה השוקים שזאת התושבת היא מיתר גם אותה תחלק התושבת לשני חלקי' שוים ‫<ref>marg. וזה יתבאר לפי הנחות זה החכם מתמונת ה' ול"ד מאמר א' ולדרוש אחר שבארנו וזולת זה המקום והוא שכאשר יצא קו מזוית משלש שוה השוקים ויחלקה לשני חצאים ב"ב נז' התושבת הנה יהיה עמוד התושבת כ"א כ"ח</ref> ועל זויות נצבות | ||
| + | |- | ||
| + | |The product of the whole diagonal by the whole diagonal is the area of the outer quadrangle that is its double. | ||
| + | |style="text-align:right;"|וכפילת כל הקוטר בכל הקטר יהיה תשבורת המרובע החיצון אשר הוא כפלו | ||
| + | |- | ||
| + | |So, the [product] of its half by its whole is half the area of this [outer] quadrangle. | ||
| + | |style="text-align:right;"|לכן מרובע חציו בכולו הוא מחצית זה שהוא תשבורת המרובע הזה | ||
| + | |- | ||
| + | |Therefore, they said that when you find an equilateral quadrangle, whether it is right-angled or not, you should measure it only by measuring the diagonals, so that you will never make a mistake. | ||
| + | |style="text-align:right;"|ולכן אמרו כשתמצא מרובע שוה הצלעות בין שיהיה נצב הזויות בין שלא יהיה לא תמנה אותו כי עם מדידת האלכסונים ולא תטעה לעולם | ||
| + | |- | ||
| + | |Because it is not easy for the person to identify whether the angle is right or not, and therefore one should be careful of making a mistake. | ||
| + | |style="text-align:right;"|כי להכיר האדם הזוית הנצבת מהבלתי נצבת אינו בקלות ולכן ראוי להשמר מן הטעות | ||
|- | |- | ||
| + | !<span style=color:Green>Finding the Side</span> | ||
| | | | ||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| + | !<span style=color:Green>Square</span> | ||
| | | | ||
| − | + | |- | |
| − | |style="text-align:right;"| | + | |If the quadrangle is a square and you do not know its side, or you wish to measure it by its one diagonal: |
| + | |style="text-align:right;"|ואם היה המרובע שוה הצלעות נצב הזויות ולא ידעת צלעו או רצית למדדו מקטרו האחד | ||
| + | |- | ||
| + | |Take its square and divide it into two [equal] parts: the one part is the area of that square and the square of the part is the length of its side. | ||
| + | |style="text-align:right;"|תקח מרובעו ותחלקהו על שני חלקים והחלק האחד הוא תשבורת המרובע ההוא ושרש החלק הוא אורך צלעו | ||
|- | |- | ||
| | | | ||
| − | : | + | *Example: an equilateral right-angled quadrangle ABGD [= square], the square of its diagonal is 200. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|דמיון מרובע אשר עליו אבג"ד שוה הצלעות נצב הזויות והיה מרובע קטרו ר‫' |
|- | |- | ||
| | | | ||
| − | : | + | :Divide the 200 into two parts, the one part is 100 and this is the area of the square. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{\frac{200}{2}=100}}</math> |
| + | |style="text-align:right;"|תחלק הר' לשני חלקים יהיה החלק הא' ק' והוא תשבורת המרובע | ||
|- | |- | ||
| | | | ||
| − | : | + | :The root of 100, which is 10, is the length of its side. |
| − | : | + | :<math>\scriptstyle{\color{blue}{\sqrt{100}=10}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ושרש הק' שהם י' הם אורך צלעו |
|- | |- | ||
| + | !<span style=color:Green>Rectangle</span> | ||
| | | | ||
| − | : | + | |- |
| − | |style="text-align:right;"| | + | |If it is not equilateral, but it is right-angled, when its diagonal and one of the sides are known to you and you wish to measure it by these two: |
| + | |style="text-align:right;"|וכן אם אינו שוה הצלעות אבל הוא נצב הזויות יהיה הקטר ידוע אליך והצלע האחד ורצית למדדו משתי אלה | ||
| + | |- | ||
| + | |Take the square of the diagonal and subtract it from the square of the known side; the remainder is the square of the remaining side. | ||
| + | |style="text-align:right;"|תקח מרובע הקטר ותגרע ממנו מרובע הצלע הידוע והנשאר הוא מרובע הצלע הנשאר | ||
| + | |- | ||
| + | |Extract its root and it is the length of the side. | ||
| + | |style="text-align:right;"|תקח שרשו והוא אורך הצלע | ||
| + | |- | ||
| + | |Multiply it by the known side and it is the area of the square. | ||
| + | |style="text-align:right;"|תכה אותו עם הצלע הידוע והוא תשבורת המרובע | ||
|- | |- | ||
| | | | ||
| − | : | + | *Example: rectangle ABGD, its side AB is 10 cubits and its diagonal AG is 12 cubits. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|דמיון מרובע ארוך אשר עליו אבג"ד והיה צלע א"ב י' אמות וקטר א"ג י"ב אמות |
|- | |- | ||
| | | | ||
| − | : | + | :We take the square of the diagonal, which is 144 cubits. We subtract from it 100 cubits, which is the square of the known side that is 10 cubits; 44 cubits remain. Their root is 6 cubits and two-thirds of a cubit minus something, which is the length of the other side. |
| − | : | + | :<math>\scriptstyle{\color{blue}{\sqrt{12^2-10^2}=\sqrt{144-100}=\sqrt{44}\approx6+\frac{2}{3}}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|לקחנו מרובע הקטר והוא קמ"ד אמות גרענו ממנו ק' אמה שהוא מרובע הצלע הידוע שהוא י' אמות ונשארו מ"ד אמות ושרשם ו' אמות ושני שלישי אמה פחות משהו שהוא אורך הצלע השני |
|- | |- | ||
| | | | ||
| − | : | + | :We multiply 6 and two-thirds minus something by 10; it is 66 cubits and two-thirds minus something and this is the area of the rectangle. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{\left(6+\frac{2}{3}\right)\sdot10=66+\frac{2}{3}}}</math> |
| + | |style="text-align:right;"|הכינו הו' ושני שלישי' פחות משהו עם הי' ונהיו ס"ו אמות ושני שלישים פחות משהו והוא תשבורת המרובע | ||
| + | |- | ||
| + | |The proof of these two measurements: it is known that the diagonal divides these two quadrangles into two equal right triangles, since their sides are parallel and the diagonal is shared by both, in each triangle there is one right angle and the diagonal is the hypotenuse opposite to the right angle in each triangle. | ||
| + | |style="text-align:right;"|<big>המופת</big> על שתי ‫<ref>45r</ref>על שתי אלו המדידות ידוע שהקטר הוא חילק את שני המרובעים האלה על שני משלשים שוים נצבי הזויות להיות שהם נכוחיי הצלעות והקטר משותף בשניהם וזוית אחת בכל משלש נצבת והקטר הוא מיתר הזוית הנצבת לכל משולש מהם | ||
|- | |- | ||
| − | | | + | |It has already been clarified in geometry that [the sum of] the two squares of the sides surrounding the right angle in the right-angled triangle is equal to the square of the side that is the hypotenuse opposite to the right angle. |
| − | + | |style="text-align:right;"|וכבר התבאר בחכמת המדות שהמשולש הנצב הזוית שני המרובעים אשר יהיו מהצלעות המקיפות בזוית הנצבת שוות למרובע ההוה מהצלע שהוא מיתר לזוית הנצבת | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| − | | | + | |Therefore, when you subtract one of them from the [sum of] the two the other remains and its root is the required. |
| − | + | |style="text-align:right;"|א"כ כשתגרע מהשוה לשנים האחד ישאר השני ושרשו הוא המבוקש | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| − | | | + | |The result of multiplying it by the other that surrounds the right angle is the area of that quadrangle. |
| − | + | |style="text-align:right;"|הנולד מכפילתו בחברו אשר יקיף עמו בזוית הנצבת הוא תשבורת המרובע ההוא | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| − | | | + | |If the area of the quadrangle is known to you and you also know one of its side, but you do not know its other side: |
| − | + | |style="text-align:right;"|ואם היתה תשבורת המרובע ידועה לך וידעת גם צלעו האחד ולא ידעת גם צלעו השני | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| − | | | + | |Divide the area of the [rectangle] by the known side and you receive the other unknown side. |
| − | + | |style="text-align:right;"|תחלק תשבורת המרובע על הצלע הידוע ויצא לך הצלע האחר הבלתי ידוע | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | : | + | *Example: a [right-angled] quadrangle, its area is 100 cubits and one of its sides is 10 cubits. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|דמיון היה מרובע תשברתו ק' אמה וצלעו האחד י' אמה |
|- | |- | ||
| | | | ||
| − | :: | + | :Divide its area, which is 100 cubits by 10; you receive 10 and from this you know that its other side is also 10 cubits and the quadrangle is equilateral. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{\frac{100}{10}=10}}</math> |
| + | |style="text-align:right;"|תחלק תשברתו שהוא הק' אמה על י' ויצא לך החלק י' ומזה ידעת שגם צלעו האחר י' אמות והמרובע הזה שוה הצלעות | ||
|- | |- | ||
| | | | ||
| − | + | *Likewise if its area is 100 cubits and one of its sides is 20 cubits. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכן אם היה תשברתו ק' אמה וצלעו הא' כ' אמה |
|- | |- | ||
| − | |||
| | | | ||
| + | :Divide 100 by 20; you receive 5, which is its other side, and from this you know that it is a parallelogram that is called rectangle. | ||
| + | :<math>\scriptstyle{\color{blue}{\frac{100}{20}=5}}</math> | ||
| + | |style="text-align:right;"|תחלק הק' על הכ' ויצא לך החלק ה' והוא צלעו האחר ומזה ידעת שהוא שטח נכחי הצלעות אשר יקרא מרובע ארוך | ||
|- | |- | ||
| − | | | + | |Proof: the area is a number that is generated from the product of the parts of one side by the parts of the other side. |
| − | + | |style="text-align:right;"|<big>המופת</big> על זה כי התשבורת הוא מספר נולד מכפילת חלקי הצלע האחד בחלקי הצלע האחר | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| − | | | + | |The number multiplied by another number is found in this product of the one number as the quantity of the other number and also the other [number] as this one. |
| − | + | |style="text-align:right;"|והמספר הנכפל במספר אחר ימצא באותה תולדה המספר האחד בכמות המספר האחר וכן האחר בזה | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| − | | | + | |So, when you know the quantity of one number and the product, if you divide the product by the quantity of the known number, we know the number that is found [in the product] as the quantity of the known number. |
| − | + | |style="text-align:right;"|וכשידעת כמות מספר האחד והתולדת אם תחלק התולדת על כמות המספר הנודע ידענו המספר אשר ימצא בכמות המספר הנודע | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| − | | | + | |The product is generated from its duplication as the times of the known number. |
| − | + | |style="text-align:right;"|ובהתקבצו כפעמי המספר הנודע נהיה התולדת | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| − | | | + | |Therefore, we divide the area of the quadrangle by its known side and this allows us to know its unknown side. |
| − | + | |style="text-align:right;"|ולכן חלקנו תשבורת המרובע על צלעו הנודע והוא יודיע לנו צלעו הבלתי נודע | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| + | !<span style=color:Green>Rhombus</span> | ||
| | | | ||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | * | + | *If the quadrangle is equilateral but not right-angled, which is called rhombus, its one diagonal is 16 cubits and its other diagonal is 12, how much is its side? |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואם היה המרובע שוה הצלעות ובלתי נצב הזויות והוא אשר יקרא מעויין והיה קטרו הא' י"ו אמה וקטרו הב' י"ב אמה כמה יהיה צלעו |
|- | |- | ||
| − | | | + | |Divide each diagonal into two [equal] parts and take the square of one part of each. |
| − | + | |style="text-align:right;"|תחלק כל קטר לשני חלקים וקח מכל אחד מרובע החלק האחד | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| − | | | + | |Sum up the two squares as one number, extract the root and it is the side of that quadrangle. |
| − | + | |style="text-align:right;"|ותחבר שני המרובעי' כאלו הם מספר אחד וקח השרש והוא צלע המרובע ההוא | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | : | + | :Example: we divide the digonal into 8 and 8. We take the square of 8, which is 64. Similarly, [we divide] the diagonal of 12 into 6 and 6. We take the square of 6, which is 36. We add the 64 to the 36; it is 100. Its root is 10 and it is the side of the quadrangle. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{\sqrt{\left(\frac{1}{2}\sdot16\right)^2+\left(\frac{1}{2}\sdot12\right)^2}\sqrt{8^2+6^2}=\sqrt{64+36}=\sqrt{100}=10}}</math> |
| + | |style="text-align:right;"|דמיון חלקנו קטר י"ו על ח' ח' ולקחנו מרובע ח' שהם ס"ד וכן קטר הי"ב על ו' ו' ולקחנו מרובע הו' שהם ל"ו חברנו הס"ד עם הל"ו ונהיו ק' ושרשו י' והוא צלע המרובע | ||
|- | |- | ||
| | | | ||
| − | : | + | :The proof of this is that each side of this quadrangle is a diagonal of the rectangle whose one side is 8 and its other side is 6, as we said in the measurement of this quadrangle. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<big>המופת</big> על זה כי כל צלע מצלעי זה המרובע יהיה קטר לשטח נכחי הצלעות נצב הזויות אשר צלעו הא' ח' וצלעו השני ו' כאשר הודענו במדידת המרובע הזה |
|- | |- | ||
| | | | ||
| − | : | + | :The square of the diagonal must be equal to [the sum of] the two squares, because it is the hypotenuse of the triangle opposite to right angle, therefore the side of the quadrangle is equal to the root of [the sum of] the two squares of half its diagonals. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ומרובע הקטר ראוי להיותו שוה לשני מרובעי כי הוא מיתר למשלש בזויתו הנצבת ולכן ישוה זה צלע המרובע לשרש ‫<ref>P1031: שני ש</ref> שני מרובעי חציי קטריו |
|- | |- | ||
| | | | ||
| − | : | + | *If one asks: a rhombus, one diagonal of which is 16 cubits and its side is 10 cubits, how much is its other diagonal? |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואם שאל השואל מעויין שקטרו האחד ‫<ref>45v</ref>שקטרו האחד י"ו אמה וצלעו י' אמה כמה קטרו האחר |
|- | |- | ||
| | | | ||
| − | : | + | :Take the square of half the known diagonal, which is 8; its square is 64. Subtract it from the square of the side, which is 100; the remainder, which is 36 is the square of half the other diagonal. Extract its root; it is 6 and this is half the diagonal. Double it; it is 12 and it is the other diagonal. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|תקח מרובע מחצית הקטר הידוע שהוא ח' ומרובעו ס"ד ותגרעהו ממרובע הצלע שהוא ק' ונשאר שהוא ל"ו הוא מרובע חצי הקטר האחר תקח שרשו והוא ו' והוא מחצית הקטר כפלהו והוא י"ב והוא הקטר האחר |
|- | |- | ||
| − | | | + | |colspan=2| |
| − | : | + | :<math>\scriptstyle{\color{blue}{2\sdot\sqrt{10^2-\left(\frac{1}{2}\sdot16\right)^2}=2\sdot\sqrt{100-8^2}=2\sdot\sqrt{100-64}=2\sdot\sqrt{36}=2\sdot6=12}}</math> |
| − | |||
|- | |- | ||
| − | + | |Proof: it is known that half of each of the two diagonals stands on the other diagonal at right angle. | |
| − | + | |style="text-align:right;"|<big>המופת</big> על זה ידוע שמחצית כל קטר משניהם עומד על הקטר האחר על זויות נצבות | |
|- | |- | ||
| − | | | + | |Half of the known diagonal with half of the unknown diagonal and the side of the quadrangle form a right-angled triangle, such that the side is the hypotenuse opposite to right angle. |
| − | + | |style="text-align:right;"|ומחצית הקטר הידוע עם מחצית הקטר הבלתי ידוע וצלע המרובע יעשו משלש נצב הזויות והצלע יהיה מיתר הזוית הנצבת | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| − | | | + | |The square of the side, which is the hypotenuse opposite to right angle, is equal to [the sum of] the squares of the two remaining sides, which are half the diagonals. |
| − | + | |style="text-align:right;"|ומרובע הצלע שהוא מיתר לזוית הנצבת ישוה לשני מרובעי שתי הצלעות הנשארות שהם חציי הקטרים | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| − | | | + | |When you subtract the square of the one side surrounding the right angle, which is half the diagonal here, from the square of the hypotenuse opposite to right angle, the square of half the other diagonal that surrounds the right angle remains and its root is half the diagonal. |
| − | + | |style="text-align:right;"|וכאשר תגרע מרובע הצלע הא' המקיף בזוית נצבת אשר הוא בזה מחצית הקטר ממרובע צלע המרובע שהוא המיתר לזוית הנצבת ישאר מרובע חצי הקטר האחר שהוא המקיף האחר לזוית הנצבת ושרשו הוא חצי הקטר | |
| − | |style="text-align:right;"|תגרע | ||
|- | |- | ||
| − | | | + | |If you double it, you find the whole diagonal. |
| − | + | |style="text-align:right;"|ואם תכפלהו תמצא כל הקטר | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| − | | | + | |As such questions you can also draw other questions and answer them. |
| − | :: | + | |style="text-align:right;"|ובכאלה השאלות תוכל להוציא גם אתה שאלות אחרות ולהשיב עליהן |
| − | |style="text-align:right;"| | + | |- |
| + | |Here comes the explanation of the measuring of various triangles, then we explain the measuring of the trapezoids, because we cannot explain the measuring of the trapezoids before the measuring of the triangles, since the triangles are basis of the trapezoids. | ||
| + | |style="text-align:right;"|ומהנה נכנס בבאור מדידת המשולשי' למיניהם אחר נבאר מדידת הנוטים כי לא נוכל לבאר מדידת הנוטים ראשונה למדידת המשולשים אחר שהמשולשים הם יסוד לנוטים | ||
| + | |- | ||
| + | |For, every trapezoid is divided into two different triangles or more. | ||
| + | |style="text-align:right;"|כי כל נוטה יחלק לשני משולשים מתחלפים או ליותר מזה | ||
| + | |- | ||
| + | |So, when you know the measuring of the different triangles, you can easily gain the knowledge of measuring the trapezoids. | ||
| + | |style="text-align:right;"|וכאשר תדע מדידת המשולשים למיניהם יגיע לך בקלות ידיעת מדידת הנוטים | ||
|- | |- | ||
| | | | ||
| − | :: | + | |
| − | |style="text-align:right;"| | + | ==== Chapter Three on the Measuring of the Various Triangles ==== |
| + | |||
| + | |style="width: 45%; text-align:right;"|<big>הפרק השלישי</big> במדידת המשולשים למיניהם | ||
| + | |- | ||
| + | |You already know that the triangles are divided into equilateral, isosceles, and scalan triangles. | ||
| + | |style="text-align:right;"|כבר ידעת שהמשולשים נחלקו לשוי הצלעות ולשוי השוקים ולמתחלפי הצלעות | ||
| + | |- | ||
| + | |We will first explain the methods of the equilateral triangle, because the height falls in it on half the base, for any side you wish to extract; then we will discuss the other triangles. | ||
| + | |style="text-align:right;"|ונקדים לבאר תחלה דרכי המשלש השוה הצלעות כי בו יפול העמוד במחצית התושבת בכל צלע שתרצה להוציאו אחר נדבר על המשלשים האחרים | ||
| + | |- | ||
| + | |We first say that the common thing for the measuring of all the triangles is that you multiply the height by half the base, or the whole base by half the height and the result is the area of the required triangle. | ||
| + | |style="text-align:right;"|ונאמר בתחלה כי הדבר הכולל לכל המשלשים במדידתם הוא שתכפול העמוד במחצית התושבת או כל התושבת במחצית העמוד וההוה הוא תשבורת המשלש המבוקש | ||
| + | |- | ||
| + | |Therefore one should first state how the height is found in each triangle, so that it becomes known, because with it we receive the unknown area of the triangle. | ||
| + | |style="text-align:right;"|ומפני זה ראוי להודיע בתחלה איך יצא העמוד בכל משלש ומשלש עד שיהיה ידוע כי בו יגיע לנו תשבורת המשלש המוסכלת | ||
|- | |- | ||
| + | !<span style=color:Green>Equilateral triangle and isosceles triangle</span> | ||
| | | | ||
| − | : | + | |- |
| − | + | |We say that in the equilateral triangle the height always falls on half the base, since its three angles are always acute angles and its three sides are equal. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ונאמר כי במשלש השוה הצלעות לעולם יפול העמוד במחצית התושבת אחר שג' זויותיה הם חדות לעולם וג' צלעותיה שוות |
| + | |- | ||
| + | |By height I mean the line that falls from the vertex at the top of the triangle to its base at right angle, which is called the height of the shape. | ||
| + | |style="text-align:right;"|וארצה בעמוד הקו הנופל מזוית ראש המשלש אשר יקרא גובה התמונה על תושבתה על זויות נצבות | ||
|- | |- | ||
| | | | ||
| − | * | + | *Example: <math>\scriptstyle\triangle ABG</math> is equilateral and the height that is drawn from the top of the triangle, which is point A, to its base falls on point D. I say that point D is the midpoint of the base. |
| − | |style="text-align:right;"|<big> | + | |style="text-align:right;"|המשל בזה משלש אב"ג שוה הצלעות ועמוד אשר יצא מראש המשלש אשר הוא נקדת א' על תושבתה יפול בנקדת ד' ואומר שנקדת ד' היא מחצית התושבת |
| + | |- | ||
| + | |Proof: the height divides the triangle into two triangles, two sides of each of these triangles are equal to two sides of the other, each to its corresponding: | ||
| + | |style="text-align:right;"|<big>המופת</big> בזה העמוד חולק את המשלש לשני משלשים אשר שתי צלעות כל משולש ממנו שוות לשתי הצלעות מהאחר כל אחד ‫<ref>46r</ref>לגילו | ||
|- | |- | ||
| | | | ||
| − | : | + | ::<math>\scriptstyle AG=AB</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|צלע א"ג שוה לצלע א"ב |
|- | |- | ||
| | | | ||
| − | : | + | ::AD is a common side of both. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ועמוד א"ד צלע משותף לשתיהן |
|- | |- | ||
| | | | ||
| − | : | + | :<math>\scriptstyle\measuredangle ADB=\measuredangle ADG</math>, because both are right angles. |
| − | + | |style="text-align:right;"|וזוית אד"ב שוה לזוית אד"ג כי שתיהן נצבות | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | : | + | :Therefore, <math>\scriptstyle DG=DB</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אם כן צלע ג"ד שוה לצלע ד"ב |
|- | |- | ||
| | | | ||
| − | : | + | :<math>\scriptstyle DG+DB</math> are the whole base, so each of them is half the base. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וצלע ג"ד וד"ב הם מחוברים הם כל התושבת אם כן כל אחת מהן היא מחצית התושבת |
| + | |- | ||
| + | |The proof that the area of every triangle is the product of the height by half the base or of the whole base by half the height, whether it is an equilateral triangle, or an isosceles triangle, whose height is drawn on its different side: | ||
| + | |style="text-align:right;"|<big>והמופת</big> אשר תשבורת כל משלש הוא כפילת העמוד בחצי תושבת או כל התושבת בחצי העמוד אם במשולש שוה הצלעות או במשלש שוה השוקים אשר הוצאת עמודו על צלעו המתחלפת | ||
| + | |- | ||
| + | |The same proof that applies to the equilateral triangle, applies to the isosceles triangle, whose height is drawn on its different side. | ||
| + | |style="text-align:right;"|הוא זה כי המופת אשר הורה במשלש שוה הצלעות הוא בעצמו יורה זה על שוה השוקים כאשר הוצאת עמודו על צלעו המתחלפת | ||
| + | |- | ||
| + | |Because, when you draw the height on half the base, then you draw a line at the end of the base parallel to the height and you draw also a line from the top of the height parallel to half the base, a rectangle is formed, whose one side is the height and its other side is half the base. | ||
| + | |style="text-align:right;"|כי כאשר הוצאת העמוד על מחצית התושבת אח"כ הוצאת קו על קצה התושבת נכחי אל העמוד עוד הוצאת קו מראש העמוד הנכחי אל מחצית התושבת כבר נהיה שטח נכחי הצלעות אשר צלעו האחת העמוד וצלעו האחר מחצית התושבת | ||
| + | |- | ||
| + | |[The area of] this surface is equal to [the area of] the mentioned triangle, for the side of the mentioned triangle is a diagonal of the mentioned rectangle that divides it into two equal triangles, such that one triangle is half the whole mentioned triangle. | ||
| + | |style="text-align:right;"|והשטח הזה שוה למשלש הנזכר כי צלע המשלש הנז' הוא קטר לנכחי הצלעות הנז' ומחלק אותו לשני המשלשים שוים והמשולש האחד הוא מחצית כל המשלש הנזכר | ||
| + | |- | ||
| + | |Therefore the whole rectangle divided into two [equal] triangles is equal to the whole mentioned triangle. | ||
| + | |style="text-align:right;"|אם כן כל שטח הנכחי הצלעות אשר הוא נחלק לשני משולשים הוא שוה לכל המשלש הנז‫' | ||
| + | |- | ||
| + | |The area of the rectangle is the product of its one side by its other side. | ||
| + | |style="text-align:right;"|ותשבורת השטח הנכחי הצלעות הוא כפילת צלעו האחת בצלעו האחרת | ||
| + | |- | ||
| + | |Its one side is the height of the triangle and its other side is half the base of the triangle. | ||
| + | |style="text-align:right;"|וצלעו האחת היא עמוד המשלש וצלעו האחרת היא מחצית תושבת המשלש | ||
| + | |- | ||
| + | |Hence, the area of the triangle is the product of the height by half its base, since it is equal to [the area of] the rectangle. | ||
| + | |style="text-align:right;"|אם כן תשבורת המשלש הוא כפילת העמוד בחצי תושבתו אחר שהוא שוה לשטח הנכחי הצלעות | ||
|- | |- | ||
| | | | ||
| − | : | + | *Example: <math>\scriptstyle\triangle ABG</math> is equilateral or isosceles, and its height is line AD. We draw from the base of this triangle at point B line BH parallel to line AD and from point H we draw line AH parallel to line DB. I say that rectangle ADHB is equal to <math>\scriptstyle\triangle ABG</math>. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|המשל בזה משלש אב"ג הוא שוה הצלעות או השוקים ועמודו הוא קו א"ד והוצאנו על קצה מחצית תושבת המשלש הזה קו ב"ה על נקדת ב' נכחי לקו א"ד ועל נקדת ה' הוצאנו קו א"ה נכחי לקו ד"ב הנה אומר כי שטח א"דה"ב הנכחיי הצלעו' שוה למשלש אב"ג |
|- | |- | ||
| | | | ||
| − | : | + | :Proof: <math>\scriptstyle\measuredangle AHB=\measuredangle ADB</math>, because both are right angles. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<big>המופת</big> בזה כי זוית אה"ב שוה לזוית אד"ב כי שתיהן נצבות |
|- | |- | ||
| | | | ||
| − | : | + | :The diagonal, which is side AB of the triangle that divides the surface into two equal triangles, because it is a straight line that falls on two parallel lines, forms the two alternate angles equal. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והקטר שהוא צלע המשלש שהוא א"ב חלק את השטח לשני משלשים שוים אחר שהוא קו ישר ונפל על שני קוים נכוחיים שם את שתי הזויות המומרות שוות |
|- | |- | ||
| − | |||
| | | | ||
| + | ::Therefore, <math>\scriptstyle\measuredangle BD=\measuredangle BAH</math> | ||
| + | |style="text-align:right;"|אם כן זוית ב"ד היא שוה לזוית בא"ה | ||
|- | |- | ||
| | | | ||
| − | + | ::The diagonal AB is a common [side]. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וקטר א"ב משותף |
|- | |- | ||
| | | | ||
| − | : | + | :Hence, <math>\scriptstyle\triangle HAB=\triangle ABD</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אם כן משלש הא"ב שוה למשלש אב"ד |
|- | |- | ||
| | | | ||
| − | : | + | :<math>\scriptstyle\triangle ABD</math> is half the rectangle HADB and it is half <math>\scriptstyle\triangle ABG</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ומשלש אב"ד הוא חצי שטח הנכחי הצלעות שהוא שטח ה"אד"ב והוא חצי משלש אב"ג |
|- | |- | ||
| | | | ||
| − | : | + | :So, the rectangle HABD is equal to <math>\scriptstyle\triangle ABG</math>. |
| − | + | |style="text-align:right;"|אם כן שטח ה"אב"ד שוה למשולש אב"ג | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | :: | + | :The area of the rectangle is the product of its one by its other side. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ושטח נכחי הצלעות הנצב הזויות כפילת צלעו האחת בצלעו האחרת היא תשברתו |
| + | |- | ||
| + | | | ||
| + | :Therefore, the area of <math>\scriptstyle\triangle ABG</math> is also the product of the one side of the rectangle, which is the height of the triangle, by its other side, which is half the base of the triangle. | ||
| + | |style="text-align:right;"|אם כן גם תשבורת משלש אב"ג הוא כפילת צלע נכחי הצלעות האחת שהוא עמוד המשלש בצלעו האחרת שהוא מחצית תושבת המשולש | ||
|- | |- | ||
| | | | ||
| − | : | + | :Q.E.D. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וזמש"ל |
|- | |- | ||
| − | | | + | |The proof that by multiplying half the height by the whole base we find the area is, if you want, from the known rule that for every number, the product of its half by a whole other number is as the product of its whole by half that number. |
| − | + | |style="text-align:right;"|<big>ואם</big> המופת שבכפילת מחצית העמוד על ‫<ref>46v</ref>על כל התושבת נמצא התשבורת הוא זה אם תרצה מהכלל הידוע שכל חשבון כפולת חציו בחשבון אחר כלו הוא ככפולת כלו כמחצית אותו החשבון | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| − | | | + | |If you want, we provide proof in geometry: |
| − | : | + | |style="text-align:right;"|ואם תרצה נביא מופת מחכמת המדות והוא זה |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | : | + | :<math>\scriptstyle\triangle ABG</math> is equilateral. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|משלש אב"ג שוה הצלעות |
|- | |- | ||
| | | | ||
| − | : | + | :We draw a height from point A of it to base BG, which is line AD. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ונוציא מנקדת א' ממנו עמוד אל תושבת ב"ג והוא קו א"ד |
|- | |- | ||
| | | | ||
| − | : | + | :We mark point H in its middle. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ונסמן במחציתו נקדת ה‫' |
|- | |- | ||
| | | | ||
| − | :: | + | :We draw two adjacent straight lines equal to base BG that are HZ and HC. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle HZ+HC=BG</math> |
| + | |style="text-align:right;"|ונוציא מנקדת ה' שני קוים דבקים על יושר נכוחיים ושוים לתושבת ב"ג והם ה"ז ה"ח | ||
|- | |- | ||
| | | | ||
| − | : | + | :We join lines CB and ZG. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ונגיע קוי ח"ב ז"ג |
|- | |- | ||
| | | | ||
| − | : | + | :I say that rectangle BGZC is equal to <math>\scriptstyle\triangle ABG</math>. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|הנה אומר כי שטח ב"ג ז"ח הנכחיי הצלעות שוה למשלש אב"ג |
|- | |- | ||
| | | | ||
| − | :: | + | :The proof: |
| − | |style="text-align:right;"| | + | :Trapezoid TKBG is shared by surface CGZC and triangle ABG |
| + | |style="text-align:right;"|<big>המופת</big> כי נוטה ט"כב"ג משותף לשטח ב"גז"ח ולמשלש אב"ג | ||
|- | |- | ||
| | | | ||
| − | :: | + | :What is left: <math>\scriptstyle\triangle CTB=\triangle ATH</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ונשאר משלש חט"ב כמשלש אט"ה |
|- | |- | ||
| | | | ||
| − | :: | + | :Because: |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle\measuredangle CTB=\measuredangle HTA</math> |
| + | |style="text-align:right;"|כי זוית חט"ב שוה לזוית הט"א | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle | + | ::<math>\scriptstyle\measuredangle BCT=\measuredangle AHT</math> |
| − | | | + | |style="text-align:right;"|וזוית אה"ט שוה לזוית בח"ט |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle AH=BC</math> because both are half the height. |
| + | |style="text-align:right;"|וצלע א"ה שוה לצלע ב"ח כי שתיהם מחצית העמוד | ||
|- | |- | ||
| | | | ||
| − | + | :Therefore: <math>\scriptstyle\triangle CTB=\triangle ATH</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אם כן משלש חט"ב שוה למשלש אט"ה |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Also: <math>\scriptstyle\triangle KZG=\triangle AKH</math> |
| + | |style="text-align:right;"|וכן משלש כז"ג שוה למשלש אכ"ה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::The procedure is the same. |
| + | |style="text-align:right;"|וההנהגה אחת | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :So, trapezoid TKBG + <math>\scriptstyle\triangle CTB+\triangle KZG</math>, which is the whole rectangle, is equal to trapezoid TKBG + <math>\scriptstyle\triangle ATH+\triangle AKH</math>, which is the whole <math>\scriptstyle\triangle ABG</math>. |
| + | |style="text-align:right;"|א"כ נוטה ט"כב"ג עם שני משולשי חט"ב כז"ג שהם כל השטח נכחי הצלעות שוה לנוטה ט"כב"ג עם שני משולשי אט"ה אכ"ה שהם כל משלש אב"ג | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Therefore: <math>\scriptstyle\triangle ABG</math> is equal to rectangle BGZC. |
| + | |style="text-align:right;"|אם כן משלש אב"ג שוה לשטח נכחי הצלעות ב"גז"ח | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :The area of the rectangle is the product of its one side, which is equal to half the height of the triangle, by its other side, which is equal to the whole base of the triangle. |
| + | |style="text-align:right;"|ותשבורת השטח הנכחי הצלעות הוא הכאת צלעו האחד שהוא שוה למחצית העמוד של המשולש בצלעו האחר שהוא שוה לכל תושבת המשולש | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Hence, the area of the triangle that is equal to it is also the product of half the height by the whole base. |
| + | |style="text-align:right;"|אם כן גם תשבורת המשולש שהוא שווה לו הוא הכאת מחצית העמוד בכל התושבת | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Q.E.D. |
| + | |style="text-align:right;"|וזה מש"ל | ||
|- | |- | ||
| − | | | + | |This same proof applies to the isosceles triangle when you draw the height on its different side. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכן זה המופת בעצמו יורה על משלש שוה השוקים כאשר הוצאת העמוד על צלעו המתחלפת |
|- | |- | ||
| − | | | + | |You already know that when you have an equilateral triangle or an isosceles triangle, you multiply the height by half the base, or vice versa, then you find the area. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|הנה כבר ידעת שכשיבא בידך הן משלש שוה הצלעות הן שוה השוקים תכפול העמוד במחצי' התושבת או ההפך ותמצא התשבורת |
|- | |- | ||
| − | | | + | |In order to find the height from the side in an equilateral triangle or an isosceles triangle, do as follows: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ולדעת העמוד מהצלע הן במשלש שוה הצלעות או במשולש שוה השוקים תעשה כן |
|- | |- | ||
| − | | | + | |Take the square of half the base, subtract it from the square of the side, then extract the root of what remains and this is the height. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|תקח מרובע חצי התושבת ותגרעהו ממרובע הצלע ואשר <sup>ישאר</sup> יקח שרשו והוא העמוד |
|- | |- | ||
| − | | | + | |Proof: the side of the triangle is the hypotenuse opposite to the right angle surrounded by the height and half the base. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<big>המופת</big> על זה כי צלע המשולש הוא מיתר הזוית הנצבת אשר יקיפו בה העמוד ומחצית התושבת |
|- | |- | ||
| − | | | + | |It has already been clarified in geometry that the square of the hypotenuse opposite to the right angle is equal to [the sum of] the squares of the two sides surrounding it. Therefore, when you subtract the square of one side from the square of the hypotenuse opposite to the right angle, then extract the root of the remainder, you find the other side. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והתבאר בחכמת השעור כי מרובע הצלע אשר היא מיתר הזוית הנצבת [שוה לשני מרובעי צלעות המקיפות בה לכן כשתגרע מרבע הצלע האחת ממרובע המיתר הזוית הנצבת‫]‫<ref>P1031 om. marg.: שוה לב' המרבעי' ההוי' מהב' צלעו' הנשארי' הוי' מרבע הצלע האחד ממרבע' המיתר והקו</ref> ותקח שרש הנשאר תמצא את צלע האחרת |
|- | |- | ||
| − | | | + | |However, the geometricians gave other rules of measuring the equilateral triangle, in order to make it easier for the one who calculates, and said: always take from 15 [parts of] the side its 13 [parts] and this is the height; or square the side, subtract its quarter from it, then extract the root of the three-quarters and this is the height of this triangle. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אולם בעלי השעור נתנו כללים אחרים במדידת המשולש השוה הצלעות כדי להקל על המחשב ואמרו לעולם תקח מט"ו בצלעו י"ג והוא העמוד או תהיה מרבע את הצלע והוצא ממנו הרביעית וקח [שרש השלש רביעיות]‫<ref>P1031: שתי הרביעיות הג' </ref>והוא עמוד המשולש הזה |
|- | |- | ||
| − | | | + | |These rules and their like are derived from the aforementioned proof. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואלה הכללים וכדומה להם יצאו מהמופת הנז‫' |
|- | |- | ||
| + | |What we have said regarding the isosceles triangle is when you draw its height one its different side, but if you draw it on one of its legs, know that it has two other rules that we will explain when we will discuss the scalene triangle. | ||
| + | |style="text-align:right;"|ומה שאמרנו בענין משלש שוה השוקים הוא כשהוצאת עמודו על צלעו המתחלפת אבל אם הוצאת אותו על צלע אחד מצלעותיו דע שיש לו שני משפטים אחרים נבארם כשנבאר ‫<ref>47r</ref>משפטי המשלש שמתחלף הצלעות | ||
| + | |- | ||
| + | !<span style=color:Green>Scalene triangle</span> | ||
| | | | ||
| − | |||
|- | |- | ||
| − | | | + | |If we wish to measure a scalene triangle, we should extract its height on its side first and to know its distance from one of the sides, for the height that we extract in the equilateral triangle and in the isosceles triangle always falls on the middle of the base, yet this height that is drawn in the scalene triangle does not fall on the middle [of the base] at all, but it is far from one side and close to the other side. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אם נרצה לשער המשלש המתחלף הצלעות תחלה ראוי להוציא עמודו על צלעו המתחלפת ולדעת מרחקו מאחת הצלעות כי העמוד אשר הוצאנו במשלש השוה הצלעות ובמשולש שוה השוקים הוא נופל במחצית התושבת לעולם אבל אין העמוד הזה היוצא במשולש המתחלף הצלעות יוצא על המחצית כלל אבל הוא רחוק מהצלע האחת והוא קרוב אל הצלע השנית |
| + | |- | ||
| + | |We call its distance from the first side "the close distance" and we call its distance from the far side "the far distance". | ||
| + | |style="text-align:right;"|ומרחקו מהצלע האחת אנו קוראים אותו המרחק הקרוב ומרחקו מהצלע הרחוק אנו קוראים אותו המרחק הרחוק | ||
|- | |- | ||
| − | | | + | |After we know the position of the height on one of the sides, we can then announce the length of its height. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואחר שנדע גבול מעמדו באחת מהצלעות אז נביא להודיע אורך עמודו |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Let us say: <math>\scriptstyle\triangle ABG</math> is a scalene triangle, whose side AB is 20 cubits, side AG is 18 cubits, and side GB is, on which we draw the height, which is the base, is 16 cubits. |
| + | |style="text-align:right;"|ונאמר משלש אב"ג מתחלף הצלעות אשר צלע א"ב ממנו עשרים אמה וצלע א"ג ממנו י"ח אמה וצלע ג"ב ממנו אשר עליו נוציא את העמוד והוא התושבת י"ו אמה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :We wish to know the distance of the height from its position to side AG, which is the short side: |
| + | |style="text-align:right;"|ונרצה לדעת גבול מעומד העמוד מצלע א"ג אשר הוא הקצר | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::We already know that the length of this side is 18 cubits. |
| + | |style="text-align:right;"|וכבר ידענו שאורך הצלע הזה י"ו אמה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::We take its square, it is 324. |
| + | |style="text-align:right;"|נקח מרובעו הוא שכ"ד | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::We add to it the square of the base, which is 256; the result is 580. |
| + | |style="text-align:right;"|ונחבר אליו מרובע התושבת שהוא רנ"ו ויהיו תק"ף | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::Then, we subtract the square of AB, which is the longer side, from 580; we already know that it is 20, and its square is 400; we subtract it from 580; the remainder is 180. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אחר נגרע מן תק"ף מרובע א"ב אשר הוא הצלע הארוך וכבר ידענו שהוא כ' ומרובעו הוא ת' נגרעם מן תק"ף נשארו ק"פ |
|- | |- | ||
| | | | ||
| − | :: | + | ::We take its half; it is 90. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|נקח מחציתם והם צ‫' |
|- | |- | ||
| | | | ||
| − | :: | + | ::We divide it by the base, whose length is 16; the first part is 5, one-half, and an eighth; and this is the distance on the base from the position of the height to the short side. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ונחלק אותם על התושבת שארכה י"ו ונהיה החלק הא' ה' וחצי ושמינית וזהו גבול מעמד העמוד מן הצלע הקצר על התושבת |
|- | |- | ||
| − | | | + | |colspan=2| |
| − | :: | + | ::<math>\scriptstyle{\color{blue}{\frac{\frac{1}{2}\sdot\left[\left(18^2+16^2\right)-20^2\right]}{16}=\frac{\frac{1}{2}\sdot\left[\left(324+256\right)-400\right]}{16}=\frac{\frac{1}{2}\sdot\left(580-400\right)}{16}=\frac{\frac{1}{2}\sdot180}{16}=\frac{90}{16}=5+\frac{1}{2}+\frac{1}{8}}}</math> |
| − | |||
|- | |- | ||
| | | | ||
| − | :: | + | :We wish to know the distance from its position to the long side: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואם רצינו לדעת גבול מעמדו מן הצלע הארוך |
|- | |- | ||
| | | | ||
| − | :: | + | ::We take its square, which is 400, and add it to the square of the base, which is 256; together it is 656. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|נקח מרובעו שהוא ת' ונחבר אותו עם מרובע התושבת שהוא רנ"ו ושניהם תרנ"ו |
|- | |- | ||
| | | | ||
| − | :: | + | ::We subtract from it the square of the short side, which is 324; the remainder is 332. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|נגרע מאלה מרובע הצלע הקצר שהוא שכ"ד נשארו של"ב |
|- | |- | ||
| | | | ||
| − | :: | + | ::We take its half; it is 166. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|נקח מחציתם והם קס"ו |
|- | |- | ||
| | | | ||
| − | :: | + | ::We divide it by the base, which is 16; the result is 10, one-third, and one part of 24; and this is the distance from the position of the height on the base to the long side. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ונחלקם על התושבת שהם י"ו ונהיה י' ושליש וחלק אחד מכ"ד וזהו גבול מעמד העמוד מן הצלע הארוך על התושבת |
|- | |- | ||
| − | | | + | |colspan=2| |
| − | :: | + | ::<math>\scriptstyle{\color{blue}{\frac{\frac{1}{2}\sdot\left[\left(20^2+16^2\right)-18^2\right]}{16}=\frac{\frac{1}{2}\sdot\left[\left(400+256\right)-324\right]}{16}=\frac{\frac{1}{2}\sdot\left(656-324\right)}{16}=\frac{\frac{1}{2}\sdot332}{16}=\frac{166}{16}=10+\frac{1}{3}+\frac{1}{24}}}</math> |
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :We give another example of a scalene triangle with integers, i.e. that the distance of the height from its position on the base is an integer without a fraction. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|עוד נמשיל במשלש מתחלף הצלעות משל אחד על חשבון שלם ר"ל שיצא גבול מעמד עמודו על התושבת באחדים שלמים מבלתי חלק |
|- | |- | ||
| | | | ||
| − | :: | + | :We say: <math>\scriptstyle\triangle ABG</math>, whose side AG is 13, side AB is 15, and side AB that is the base is 14. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ונאמר משלש אב"ג אשר צלע א"ג ממנו י"ג וצלע א"ב ממנו ט"ו וצלע ב"ג ממנו אשר הוא התושבת י"ד |
|- | |- | ||
| | | | ||
| − | :: | + | :We wish to know the distance of the height from its position on the base to the short side, which is AG: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ורצינו לדעת גבול מעמד העמוד על התושבת מהצלע הקצר שהוא א"ג ממנו |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::We add the square of the base to the square of this side; the result is 365. |
| + | |style="text-align:right;"|חברנו אל מרובע הצלע הזה מרובע התושבת ונהיו שס"ה | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::We subtract from it the square of the long side, which is 225; the remainder is 140. |
| − | | | + | |style="text-align:right;"|גרענו מזה המרובע מהצלע הארוך אשר הוא רכ"ה ונשארו ק"מ |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::We take its half; it is 70. |
| + | |style="text-align:right;"|לקחנו חצים והם ע‫' | ||
|- | |- | ||
| | | | ||
| − | + | ::We divide it by 14, which is the base; the first part is 5; and this is the distance from the position of the height to the short side. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|חלקנום על י"ד שהוא חותך התושבת והיה החלק האחד ה' וזהו גבול מעמד העמוד מן הצלע הקצר |
|- | |- | ||
| − | | | + | |colspan=2| |
| − | + | ::<math>\scriptstyle{\color{blue}{\frac{\frac{1}{2}\sdot\left[\left(13^2+14^2\right)-15^2\right]}{14}=\frac{\frac{1}{2}\sdot\left(365-225\right)}{14}=\frac{\frac{1}{2}\sdot140}{14}=\frac{70}{14}=5}}</math> | |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :If you wish to know its distance from its position to the long side: |
| + | |style="text-align:right;"|ואם תרצה לדעת גבול מעמדו מן הצלע הארוך | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::We add its square to the square of the base; the result is 421. |
| + | |style="text-align:right;"|נחבר מרובעו עם מרובע התושבת ונהיו תכ"א | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::We subtract from it the square of the short side, which is 169; the remainder is 252. |
| + | |style="text-align:right;"|הוצאנו מהם מרובע הצלע הקצר שהוא קס"ט נשארו רנ"ב | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::We take its half; it is 126. |
| + | |style="text-align:right;"|לקחנו חצים והם קכ"ו | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::We divide it by 14, which is the base; the [second] part is 9; and this is the distance from the position of the height to the long side. |
| + | |style="text-align:right;"|וחלקנום על י"ד שהוא התושבת היה החלק הא' ט' וזהו גבול ‫<ref>47v</ref>מעמד העמוד מן הצלע הארוך | ||
|- | |- | ||
| − | | | + | |colspan=2| |
| − | + | ::<math>\scriptstyle{\color{blue}{\frac{\frac{1}{2}\sdot\left[\left(15^2+14^2\right)-13^2\right]}{14}=\frac{\frac{1}{2}\sdot\left(421-169\right)}{14}=\frac{\frac{1}{2}\sdot252}{14}=\frac{126}{14}=9}}</math> | |
|- | |- | ||
| − | | | + | |The proof of this has already been clarified in geometry, that the square of the side, which is opposite an acute angle, is less than the sum of the squares of the two other sides by double the rectangle that is encompassed by the whole base and the distance from the position of the height to that side. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<big>המופת</big> על זה כבר התבאר בחכמת השעור שמרובע הצלע אשר הוא מיתר זוית חדה הוא פוחת מן מרובעי שתי צלעות הנשארות בכפל שטח הנצב הזויות אשר יקיף בו התושבת כלו עם מקום מעמד העמוד מאותו הצד |
|- | |- | ||
| − | | | + | |Therefore, when you add the square of the side to the square of the base and subtract the square of the other side from this, the remainder is double the rectangle that is encompassed by the whole base and the distance from the position of the height to that side. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ולכן כאשר תחבר מרובע הצלע עם מרובע התושבת ותוציא מהם מרובע הצלע הנשאר ישאר ככפל הנצב הזויות אשר יקיף בו כל התושבת והחלק אשר יצא עליו העמוד מאותו הצד |
| + | |- | ||
| + | |Thus, we divide it by two and then divide by the base, as the sum is a double, and the result is the distance from the position of the height to that side. | ||
| + | |style="text-align:right;"|ולכן נחלק אותו לשנים והחלק האחד נחלקהו על התושבת אחרי שהסך הוא כפל ואשר יצא הוא מקום מעמד העמוד על אותו הצד | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :The example for this is the triangle we have drawn: |
| + | |style="text-align:right;"|המשל בזה המשלש אשר ציירנו | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::We know that side AG is 13 cubits and its square is 169. |
| + | |style="text-align:right;"|ידענו שצלע א"ג ממנו הוא י"ג אמה ומרובעו קס"ט | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::The base is 14 cubits and its square is 196. |
| + | |style="text-align:right;"|והתושבת י"ד אמה ומרובעה קצ"ו | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::The [sum of the] two squares is 365. |
| + | |style="text-align:right;"|ושתי המרובעים שס"ה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Side AB is 15 cubits and its square is 225. |
| + | |style="text-align:right;"|וצלע א"ב אשר הוא ט"ו אמה ומרובעו רכ"ה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::It is less than [the sum of] the squares mentioned by double the rectangle that is encompassed by GD by GB. |
| + | ::<math>\scriptstyle{\color{blue}{\left(AG^2+GB^2\right)-AB^2=2\sdot\left(GD\sdot GB\right)}}</math> | ||
| + | |style="text-align:right;"|יהיה פוחת משני המרובעים הנזכרי' בכפל השטח הנצב הזויות אשר יקיף בו ג"ד בג"ב | ||
|- | |- | ||
| − | | | + | |colspan=2| |
| − | + | ::<math>\scriptstyle{\color{blue}{\left(13^2+14^2\right)-15^2=\left(169+196\right)-225=365-225=2\sdot\left(GD\sdot GB\right)}}</math> | |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::So, when we subtract 225 from 365, 140 remains, which is double the rectangle that is encompassed by GD by GB. |
| + | ::<math>\scriptstyle{\color{blue}{\left(13^2+14^2\right)-15^2=365-225=140=2\sdot\left(GD\sdot GB\right)}}</math> | ||
| + | |style="text-align:right;"|א"כ כאשר גרענו רכ"ה משס"ה ישאר ק"מ והוא ככפל השטח הנצב הזויות אשר יקיף בו ג"ד בג"ב | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Since it is a double, we take its half, which is 70, and it is the same as the rectangle that is encompassed by GD by GB. |
| − | + | ::<math>\scriptstyle{\color{blue}{\frac{1}{2}\sdot\left[\left(AG^2+GB^2\right)-AB^2\right]=GD\sdot GB}}</math> | |
| − | + | |style="text-align:right;"|ומפני שהוא כפל לקחנו חציו והוא ע' והוא כשטח הנצב הזויו' אשר יקיף ג"ד בג"ב | |
|- | |- | ||
| | | | ||
| − | + | ::We divide it by the base, which is 14, and we know that the quotient is the distance from the position of the height to that side; this quotient is 5. | |
| − | + | |style="text-align:right;"|חלקנוהו על התושבת שהוא י"ד וידענו שהחלק האחד ממנו הוא מקום מעמד העמוד מאותו הצד והחלק הוא ה‫' | |
| − | |||
| − | |style=" | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Therefore, we know that the distance from the position of the height to that side is 5. |
| + | |style="text-align:right;"|ומזה ידענו שמרחק העמוד מאותו הצלע הוא ה‫' | ||
|- | |- | ||
| − | | | + | |After we have determined the position of the height, we can calculate its length. We do as follows: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואחר שידענו גבול מעמדו נבא לדעת ארכו וכן נעשה |
|- | |- | ||
| − | | | + | |We subtract the square of the distance of the height on the base from the square of the adjacent side, so the remainder is the square of the height. |
| − | + | |style="text-align:right;"|נגרע מרובע החלק מן התושבת אשר עומד עליו העמוד ממרובע הצלע הדבק בו ואשר ישאר הוא מרובע העמוד | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | : | + | *Example of the same triangle: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|המשל בזה המשלש |
|- | |- | ||
| | | | ||
| − | : | + | :We subtract the square of 5, which is 25, from the square of the side next to it, which is 169; 144 remains. Its root is 12 and this is the length of the height. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{\sqrt{13^2-5^2}=\sqrt{169-25}=\sqrt{144}=12}}</math> |
| + | |style="text-align:right;"|גרענו מרובע ה' שהוא כ"ה ממרובע הצלע הדבק בו‫<ref>P1031: ואשר ישאר הוא מרובע העמוד גרענו כ"ה ממרובע הצלע הדבק בו</ref> שהוא קס"ט [ונשארו קמ"ד]‫<ref>P1031: om.</ref> ושרשו י"ב והוא אורך העמוד | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Also, if we wish to find it by the other part of the base, which is 9 and its square is 81: we subtract it from the square of the side next to it, which is 225; 144 remains. Its root is 12 and this is the length of the height. |
| + | :<math>\scriptstyle{\color{blue}{\sqrt{15^2-9^2}=\sqrt{225-81}=\sqrt{144}=12}}</math> | ||
| + | |style="text-align:right;"|וכן אם רצינו לדעת אותו מהחלק האחר מהתושבת אשר הוא ט' ומרובעו פ"א גרענו אותו ממרובע הצלע הדבק בו אשר הוא רכ"ה נשארו קמ"ד ושרשו י"ב והוא אורך העמוד | ||
|- | |- | ||
| − | | | + | |Proof: the square of each side of them is equal to the square of the height and [the square of] the part of the base that is next to it, because each of them is the hypotenuse opposite to the right angle in its triangle. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<big>והמופת</big> על זה כי מרובע כל צלע מהם שוה עם מרובע העמוד והחלק מן התושבת הדבק בו מפני שהוא מיתר לזוית הנצבת כל א' במשלשו |
|- | |- | ||
| − | | | + | |For, when you draw the height, the triangle is divided into two triangles. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כי כאשר הוצאת העמוד כבר נחלק המשלש לשני משלשים |
| + | |- | ||
| + | |When you subtract the square of the part of the base from the square of the side next to it, the square of the height remains from the square of the side; and when you extract its root, you find the length of the height. | ||
| + | |style="text-align:right;"|וכאשר גרעת מרובע החלק מן התושבת וממרובע הצלע הדבק בו נשאר במרובע הצלע מרובע העמוד וכשלקחת שרשו מצאת אורך העמוד | ||
| + | |- | ||
| + | |We have explained the measurement of all types of triangles and their differences in terms of the sides. | ||
| + | |style="text-align:right;"|הנה בארנו מדידת כל המשלשים למיניהם והבדלם מצד הצלעות | ||
|- | |- | ||
| − | | | + | |Although the scalene triangles are included in these measurements. For, the isosceles triangle is necessarily either acute angled, right-angled, or obtuse angled, and likewise the scalene triangle. Yet, since they have special measures from the aspect of the angles also, we shall explain them, eventhough it is not necessary. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואעפ"י שבמדות האלה יכללו גם מתחלפי הזויות כי המשלש שוה השוקים לא ימלט מהיותו חד הזויות ואם נצב הזויות או נרוח גם ככה המתחלף הצלעות אמנם בעבור שיש להם מדות מיוחדות ‫<ref>48r</ref>גם מפני הזויות נבארם ואין זה צורך |
|- | |- | ||
| + | !<span style=color:Green>Right-angled triangle</span> | ||
| | | | ||
| − | |||
|- | |- | ||
| − | | | + | |We say concerning the right-angled triangle that there is no need to draw its height to its hypotenuse opposite to the right angle, because each of its two sides surrounding the right angle are its heights. |
| − | + | |style="text-align:right;"|ונאמר כי המשלש הנצב הזויות אין צורך להוציא עמודו על צלעו שהוא מיתר הזוית הנצבת כי כל אחת משתי צלעותיו המקיפות בזוית הנצבת הן עמודיו | |
| − | |style="text-align:right;"| | + | |- |
| + | |Therefore, if you multiply one of the mentioned sides by its other side surrounding the right angle, you find its area. | ||
| + | |style="text-align:right;"|ולכן אם תכה אחד מהצלעות הנזכרות בצלעו האחרת המקפת עמו הזוית הנצבת תמצא תשברתו | ||
|- | |- | ||
| − | | | + | |The proof of this is known from what we explained concerning the previous triangles. |
| − | + | |style="text-align:right;"|והמופת על זה ידוע ממה שבארנו במשלשים הקודמים | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| + | !<span style=color:Green>Obtuse triangle</span> | ||
| | | | ||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |Also with regard to the obtuse triangle, it is not necessary to draw its height to its side opposite to the obtuse angle, but if you wish to draw it, I will show you this way: |
| − | + | |style="text-align:right;"|עוד המשלש הנרוח הזוית אין הכרח להוציא עמודו על צלעו שהוא מיתר הזוית הנרוחת לבד אבל אם רצית להוציאו בדרך זו אשר אורה לך | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| − | | | + | |It has already been clarified in geometry that the square of side opposite to the obtuse angle exceeds [the sum of] the squares of the two sides surrounding it by twice the product of the base of this side by the line that is drawn outside [the triangle] from the end of the mentioned base to the place where the height falls. |
| − | + | |style="text-align:right;"|כבר התבאר בחכמת השעור שמרובע מיתר הזוית הנרוחת עודף על מרבעי שתי הצלעות המקיפות בה בכפל התושבת אשר עליה הצלע בקו היוצא חוצה מקצה התושבת הנזכרת עד מקום נפילת העמוד פעמים | |
| − | |||
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | * | + | *Example: <math>\scriptstyle\triangle ABG</math> is an obtuse triangle, and its <math>\scriptstyle\measuredangle AGB</math> is the obtuse angle. I say that the square of AB, which is the side opposite to the obtuse angle, exceeds [the sum of] the square of AG and GB by twice the product of GB by GD. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|המשל בזה משלש אב"ג נרוחת הזויות וזוית אג"ב ממנו היא הנרוחת אומר שמרובע א"ב שהוא מיתר הזוית הנרוחת עודף על מרובע צלע א"ג וג"ב בכפל <sup>ג"ב</sup> בג"ד פעמים |
|- | |- | ||
| | | | ||
| − | : | + | :The way to draw the height in this triangle is as follows: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ודרך הוצאת העמוד הזה במשלש הזה כך |
|- | |- | ||
| | | | ||
| − | : | + | :We define the obtuse angle as G. |
| − | + | |style="text-align:right;"|נשים הזוית הנרוחת אשר עליה ג‫' | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Side GA is 4 cubits; side BG is 13 cubits; and side AB that is opposite to the [obtuse] angle is 15 cubits. |
| + | |style="text-align:right;"|וצלע ג"א ד' אמות וצלע ב"ג י"ג אמה וצלע א"ב שהוא מיתר הזוית ט"ו אמה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :The square of 15 exceeds the square of 13 and the square of 4 that are 185 cubits by 40 cubits. |
| + | :<math>\scriptstyle{\color{blue}{15^2-\left(13^2+4^2\right)=15^2-185=40}}</math> | ||
| + | |style="text-align:right;"|ומרובע ט"ו מוסיף על מרובע י"ג ומרובע ד' אשר הם קפ"ה אמה מ' אמה | ||
|- | |- | ||
| − | | | + | |If you divide half the excess by one of the sides, you receive the distance of the height from that side. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואם תחלק מחצית התוספת על אחת מהצלעות יצא לך מרחק העמוד חוצה מן הצלע ההוא |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :For instance, we divide the half of 40, which is the excess, that is 20 by line GA, which is 4 cubits; the quotient is 5, so we know that the height falls 5 cubits from side GA, this is line GD and the height on it is AD. |
| + | :<math>\scriptstyle{\color{blue}{GD=\frac{\frac{1}{2}\sdot40}{4}=\frac{20}{4}=5}}</math> | ||
| + | |style="text-align:right;"|עד"מ חלקנו מחצית המ' אשר הוא התוספת והם כ' על קו ג"א אשר הוא ד' אמות ויצא החלק ה' וידענו מזה שהעמוד נפל חוצה מן צלע ג"א ה' אמות והוא קו ג"ד והעמוד עליה עמוד א"ד | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :If you divide it by line BG, whose length is 13 cubits, the quotient is one and seven parts of 13 and it is the distance of the height that falls outside from GB. |
| + | :<math>\scriptstyle{\color{blue}{\frac{\frac{1}{2}\sdot40}{13}=\frac{20}{13}=1+\frac{7}{13}}}</math> | ||
| + | |style="text-align:right;"|ואם תחלק אותו על קו ב"ג אשר ארכו י"ג אמה יצא החלק אחד ושבעה‫<ref>P1031: האחד שבעים ואחד</ref> חלקים מי"ג והוא מרחק העמוד היוצא חוצה מן ג"ב והעמוד עליו | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | |
| + | ==== Chapter Four on the Measuring of the Various Trapezoids ==== | ||
| + | |||
| + | |style="width:45%; text-align:right;"|<big>הפרק הרביעי</big> במדידת הנוטים למיניהם | ||
|- | |- | ||
| − | | | + | |The trapezoid is a quadrangle shape that is not a square, not a rectangle, not a rhombus, and not a parallelogram. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|הנוטה הוא תמונה בעלת ד' צלעות שאינה לא מרובע ולא נכחי הצלעות ארוך ולא מעויין ולא דומה למעויין |
|- | |- | ||
| − | | | + | |They are of many types, although they are all included in four types: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והם מינים רבים אעפ"י שכלם נכללים בד‫' |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *Among them are those whose two corresponding sides are equal, while the upper base and the bottom base are not equal but parallel [= isosceles trapezoid], like this: |
| + | |style="text-align:right;"|יש מהם ג' ששתי צלעותיו המקבילות שוות והראש והתושבת בלתי שוות אבל הם נכחיות כזה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *Among them are those whose two sides are equal, but the upper base and the bottom base are not equal and not parallel, like this: |
| + | |style="text-align:right;"|ויש מהם ששתי הצלעות שוות והראש והתושבת בלתי שוות ולא נכחיות כזה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *Among them are those whose two adjacent sides are equal to each other and so are the other two sides [= kite], like this: |
| + | |style="text-align:right;"|ויש מהם ששתי הצלעות הדבקות מהם שוות האחת לחברתה כזה וכן השתי צלעות האחרות | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *Among them are those who are acute-angled, like this: |
| + | |style="text-align:right;"|ויש מהם חד הזוית כזה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *Among them are those that none of their sides is equal to the other, like this: |
| + | |style="text-align:right;"|ויש מהם שאין צלע הא' שוה עם האחר כזה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *Among them are those that have two right angles, like this: |
| + | |style="text-align:right;"|ויש מהם נצב [שתי]‫<ref>P1031 om.</ref> הזויות כזה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *Among them are those that have one right angle, like this: |
| + | |style="text-align:right;"|ויש מהם נצב הזוית האחת כזה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *Among them are those that are obtuse-angled, like this: |
| + | |style="text-align:right;"|ויש מהם נרוח הזוית כזה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *Among them are curved shapes other than these. |
| + | |style="text-align:right;"|ויש מהם תמונות עקלקלות זולת אלו | ||
|- | |- | ||
| − | | | + | |Therefore I should give you the way we need for measuring them. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|לכן ראוי לך שאתן דרך הצריכה לנו במדידתם |
|- | |- | ||
| + | !<span style=color:Green>Isosceles trapezoid</span> | ||
| | | | ||
| − | |style="text-align:right;"| | + | |- |
| + | |I start with the trapezoid whose two sides are equal but not parallel, whose the top base and bottom base are not equal but are parallel. | ||
| + | |style="text-align:right;"|ואחל מהנוטה אשר שתי צלעותיו שוות ואינם נכוחיות והראש והתושבת בלתי שוות אבל ‫<ref>48v</ref>הם נכוחיות | ||
|- | |- | ||
| | | | ||
| − | + | *We say that if ABGD is a trapezoid, AB is 8 cubits, DG is 18 cubits, both AD and BG are 13 cubits each, and you wish to find its area. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ונאמר אם היה נוטה אבג"ד וצלע א"ב ח' אמות וצלע ד"ג י"ח אמות ושני צלעי א"ד ב"ג כל אחד מי"ג אמה ותרצה למצוא תשברתו |
| + | |- | ||
| + | |Do as follows: | ||
| + | |style="text-align:right;"|תעשה כך | ||
|- | |- | ||
| − | | | + | |Subtract the top base from the bottom base, take half the remainder, then count that much from one vertex of the bottom base and mark a dot there. |
| − | + | |style="text-align:right;"|תגרע הראש מהתושבת ומהמותר תקח מחציתו ומנה כמה הוא מזוית התושבת האחת וסמן שם נקדה | |
| − | |style="text-align:right;"|תקח | ||
|- | |- | ||
| − | | | + | |Count also that much from the other vertex of the bottom base and mark a dot there. |
| − | + | |style="text-align:right;"|עוד מנה כמה הוא מזוית התושבת האחרת וסמן שם נקדה | |
| − | |||
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | ::Example: we subtract 8 from 18; 10 remains. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|דמיון גרענו הח' מן הי"ח ונשארו י‫' |
|- | |- | ||
| | | | ||
| − | : | + | ::We take its half; it is 5. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\frac{1}{2}\sdot\left(18-8\right)=\frac{1}{2}\sdot10=5}}</math> |
| + | |style="text-align:right;"|לקחנו מחציתם והם ה‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | ::We count that much from vertex G and mark point Z there. |
| − | + | |style="text-align:right;"|מנינו כן מזוית ג' וסמננו שם נקדת ז‫' | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | : | + | ::We count also that much from vertex D and mark point H there. |
| − | |style="text-align:right;"|עוד | + | |style="text-align:right;"|עוד מנינו כן מזוית ד' וסמננו שם נקדת ה‫' |
|- | |- | ||
| | | | ||
| − | :: | + | ::We draw AH and BZ and so we know the heights of this shape. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|הגענו א"ה וב"ז וידענו עמודי זאת התמונה |
|- | |- | ||
| | | | ||
| − | :: | + | ::We wish to know the length of the heights: |
| − | : | + | |style="text-align:right;"|רצינו לדעת ארך העמודים |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | : | + | ::We multiply the side, which is 13, by itself; it is 169. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכפלנו הצלע שהם י"ג על עצמם והיו קס"ט |
|- | |- | ||
| | | | ||
| − | :: | + | ::We subtract from it 25, which is the square of 5; 144 remains. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|הוצאנו מהם כ"ה שהוא מרובע ה' נשארו קמ"ד |
|- | |- | ||
| | | | ||
| − | :: | + | ::We extract its root; it is 12. |
| − | ::<math>\scriptstyle{\color{blue}{\ | + | ::<math>\scriptstyle{\color{blue}{\sqrt{13^2-5^2}=\sqrt{169-25}=\sqrt{144}=12}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|לקחנו גדרם והם י"ב |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Hence, we know that the length of the height is 12. |
| + | |style="text-align:right;"|וידענו שארך העמוד הם י"ב | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::We add the top base to the bottom base; it is 26. |
| + | |style="text-align:right;"|חברנו הראש עם התושבת והם כ"ו | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::We take its half; it is 13. |
| + | |style="text-align:right;"|ולקחנו מחציתם והם י"ג | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::We multiply it by the height, which is 12; it is 156 and this is the area of trapezoid. |
| + | ::<math>\scriptstyle{\color{blue}{\left[\frac{1}{2}\sdot\left(8+18\right)\right]\sdot12=\left(\frac{1}{2}\sdot26\right)\sdot12=13\sdot12=156}}</math> | ||
| + | |style="text-align:right;"|וכפלנום עם העמוד שהם י"ב והיו קנ"ו וזהו תשבורת הנוטה הזה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *The proof of that: |
| + | |style="text-align:right;"|<big>המופת</big> על זה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :It is known that half the excess is on one side and its half is on the other side up to the heights that are [drawn] on them. |
| + | |style="text-align:right;"|ידוע שמחצית העודף יהיה מהצד האחד ומחציתו מהצד האחר עד שיוציאו העמודים עליהם | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*Side AD is the hypotenuse of the right angle. |
| + | |style="text-align:right;"|וצלע א"ד הוא מיתר הזוית הנצבת | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Therefore its square is equal to the square of the height with [the square of] the base of <math>\scriptstyle\triangle ADH</math> |
| + | |style="text-align:right;"|ולכן מרבעו שוה למרובע העמוד עם תושבת משלש אד"ה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*The base of <math>\scriptstyle\triangle ADH</math> is 5. |
| + | |style="text-align:right;"|ותושבת משלש אד"ה ה‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Its square is 25. |
| + | |style="text-align:right;"|ומרבעו כ"ה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Subtract is from the square of the side, which is 169; 144 remains. |
| + | |style="text-align:right;"|תגרעהו ממרובע הצלע אשר הוא קס"ט נשאר קמ"ד | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Its root is 12 and this is the length of the height. |
| + | ::<math>\scriptstyle{\color{blue}{\sqrt{13^2-5^2}=\sqrt{169-25}=\sqrt{144}=12}}</math> | ||
| + | |style="text-align:right;"|וגדרו י"ב והוא ארך העמוד | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*It is known that the area of <math>\scriptstyle\triangle ADH</math> is the product of the height by half DH. |
| + | |style="text-align:right;"|וידוע שתשבורת משלש אד"ה הוא כפילת העמוד בחצי ד"ה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*Also the area of <math>\scriptstyle\triangle BZG</math> is the product of the height by half ZG. |
| + | |style="text-align:right;"|וכן תשבורת משלש בז"ג הוא כפילת העמוד בחצי ז"ג | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::So, the area of both these triangles is the product of the height by the whole DH. |
| + | |style="text-align:right;"|אם כן תשבורת שני משולשים האלה הוא כפילת העמוד בכל תושבת ד"ה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::But, the area of the rectangle ABHZ is the product of the height by the base HZ. |
| + | |style="text-align:right;"|ותשבורת השטח הנצב הזויות והוא שטח אבה"ז הנה הוא כפילת העמוד בתושבת ה"ז | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :So, the product of DZ by the height is the area of trapezoid ABGD. |
| + | |style="text-align:right;"|אם כן כפילת ד"ז עם העמוד הוא תשבורת נוטה אבג"ד | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *This is also clarified by the proof of a different shape: |
| + | |style="text-align:right;"|ויתבאר גם כן זה בצורה אחרת מהמופת | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *We have an isosceles trapezoid; AB=GD, each is 13 cubits; AG is 6 cubits; BD is 16 cubits. We wish to know the area and the height. |
| + | |style="text-align:right;"|והוא יש לנו נוטה אבג"ד שוה השוקים וצלע א"ב שוה לצלע ג"ד וכל אחת מהן בעלת י"ג אמות וצלע א"ג ו' אמות וצלע ב"ד י"ו אמות ונרצה למצוא התשבורת והעמוד | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*I draw line AH parallel to line GD. |
| + | |style="text-align:right;"|אוציא קו נכחי א"ה לקו ג"ד | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*I draw height AZ to BD. |
| + | |style="text-align:right;"|ואוציא עמוד א"ז על קו ב"ד | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :So surface AHGD is a rectangle. |
| + | |style="text-align:right;"|אם כן שטח אהג"ד הוא נכחי הצלעות | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Therefore |
| + | :*<math>\scriptstyle AB=HD</math> | ||
| + | |style="text-align:right;"|וא"כ א"ב שוה לה"ד | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*<math>\scriptstyle GD=AH</math> |
| + | |style="text-align:right;"|וג"ד לא"ה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Since BD is 16 cubits and HD is 6 cubits, the remaining BH is 10 cubits. |
| + | ::<math>\scriptstyle{\color{blue}{BH=BD-HD=16-6=10}}</math> | ||
| + | |style="text-align:right;"|ולפי שב"ד י"ו אמו' וה"ד ו' אמו' א"כ ב"ה הנשארת תהיה י' אמות | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Since <math>\scriptstyle\triangle ABH</math> is isosceles and each of its sides is known, height AZ is also known and it is 12 cubits, as previously known. |
| + | |style="text-align:right;"|ולפי שמשלש אב"ה שוה השוקים וכל אחת מצלעותיו ידועה יהיה א"כ גם עמוד א"ז ידוע והוא י"ב אמות כאשר קדם ידיעתו | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*We halve AB and GD at points C and T. |
| + | |style="text-align:right;"|ונחלק צלעי א"ב ג"ד באמצע על נקדת ח"ט | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*We draw heights KCL, MTN to line BD. |
| + | |style="text-align:right;"|ונוציא עמוד כח"ל מט"נ על קו ב"ד | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Therefore: |
| + | :*<math>\scriptstyle\triangle AKC=\triangle BCL</math> | ||
| + | |style="text-align:right;"|אם כן משלש אכ"ח שוה למשלש בח"ל | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*<math>\scriptstyle\triangle DMT=\triangle GNT</math> |
| + | |style="text-align:right;"|ומשלש דמ"ט שוה למשלש גנ"ט | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::So, if we add the shared hexagon ACLNTD, the area of rectangle KLMN is equal to the area of trapezoid ABGD. |
| + | |style="text-align:right;"|לכן אם נוסיף בשתוף בעל שש צלעות א"ח ל"נ ט"ד ישוה שטח כ"ל מ"נ הנכחי הצלעות לנוטה אבג"ד | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::And since: |
| + | :*AK=BL | ||
| + | |style="text-align:right;"|ולפי שא"כ שוה לב"ל | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*DM=GN |
| + | |style="text-align:right;"|וד"מ שוה לג"נ | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::AK, DM are equal to BL, NG |
| + | |style="text-align:right;"|אם כן א"כ ד"מ שוים לב"ל נ"ג | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::When the shared AD, LN are added: <math>\scriptstyle KM+LN=2\sdot KM=AD+BG</math> |
| + | |style="text-align:right;"|וכשיתוסף עליהם בשתוף א"ד ל"נ יהיו שני' כ"מ ל"נ שהם שני פעמים כ"מ שוה לשני ‫<ref>49r</ref>א"ד ב"ג | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::AD, BG are known, for they are 22 cubits. |
| + | |style="text-align:right;"|ושני א"ד ב"ג ידועים כי הם כ"ב אמות | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Therefore: <math>\scriptstyle2\sdot KM=22</math> |
| + | |style="text-align:right;"|א"כ גם כ"מ פעמים כ"ב | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Hence, KM is 11 cubits. |
| + | |style="text-align:right;"|ויהיה כ"מ י"א אמות | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|גם | + | ::But, KL is 12 cubits. |
| + | |style="text-align:right;"|אבל גם כ"ל י"ב אמות | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::And trapezoid ABGD is equal to rectangle KLMN |
| + | |style="text-align:right;"|ונוטה אבג"ד שוה לשטח כ"ל מ"נ הנכחי בצלעות | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Therefore, its area is 132 cubits as the area of the rectangle. |
| + | |style="text-align:right;"|א"כ יהיה תשברתו קל"ב אמות כתשבורת הנכחי הצלעות | ||
|- | |- | ||
| + | !<span style=color:Green>Acute trapezoid</span> | ||
| | | | ||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *If you wish to measure an acute trapezoid, such as trapezoid ABGD, whose angle B is acute, AB is 13 cubits, GD is 20 cubits, AD is 6 cubits, and BG is 27 cubits. You wish to find the area and the height. |
| + | |style="text-align:right;"|ואם רצית למדוד נוטה חד הזוית כגון נוטה אבג"ד והזוית אשר אצל ב' חדה וצלע א"ב י"ג אמות וצלע ג"ד כ' אמות וצלע א"ד ו' אמות וצלע ב"ג כ"ז אמות ותרצה למצוא התשבורת והעמוד | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Do as follows: |
| + | |style="text-align:right;"|כך תעשה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Subtract 6 cubits from 27 cubits; 21 cubits remain. |
| + | |style="text-align:right;"|תגרע הו' אמות מן הכ"ז אמות וישארו כ"א אמות | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Since the sides of the acute triangle are known - 13, 21, 20 - the length of the height AZ is also known, which is 12 cubits, as we knew it previously. |
| + | |style="text-align:right;"|ובהיות צלעות משלש החד הזויות ידועות והם י"ג וכ"א וכ' יודע גם ארך א"ז העמוד שהוא י"ב אמות כמו שידענו זה מקודם | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Add 6 cubits to 27 cubits; its half is 16½. |
| + | |style="text-align:right;"|ותחבר עם הו' אמות כ"ז אמות ויהיה מחציתו י"ו אמות וחצי | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Multiply it by the height; it is 198 and this is the area of the trapezoid. |
| + | ::<math>\scriptstyle{\color{blue}{\left[\frac{1}{2}\sdot\left(27+6\right)\right]\sdot12=\left(16+\frac{1}{2}\right)\sdot12=198}}</math> | ||
| + | |style="text-align:right;"|כפלם עם העמוד ויהיו קצ"ח והם תשבורת הנוטה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *The proof of that: |
| + | |style="text-align:right;"|<big>והמופת</big> על זה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :I draw line AH parallel to line GD. |
| + | |style="text-align:right;"|אוציא קו א"ה נכחי לקו ג"ד | ||
|- | |- | ||
| | | | ||
| − | + | :I draw height AZ. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואוציא א"ז עמוד |
|- | |- | ||
| | | | ||
| − | : | + | :Since AH is 20 cubits and GH is 6 cubits, the remaining BH is 21 cubits. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ולפי שא"ה כ' אמות וג"ה ו' אמות אם כן ב"ה הנשאר כ"א אמות |
|- | |- | ||
| | | | ||
| − | : | + | :Since line AZ, which is the height of the acute triangle ABH, is 12 cubits; lines AB and GD are halved at points C and T; BDL is the height from TN also, as we have shown concerning the shape that precedes this one; it is clear that trapezoid ABGD is equal to the rectangle KLMN. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ולפי שקו א"ז העמוד במשלש אב"ה החד הזויות הוא י"ב אמות וצלעי א"ב ג"ד נחלקים באמצע על נקדת ח' וט' ובח"ל הוא העמוד גם מט"נ כמו שהראנו בצורה הזאת הקודמת לזה יראה שנוטה אבג"ד שוה לשטח כ"ל מ"נ נכחי הצלעות |
|- | |- | ||
| | | | ||
| − | : | + | ::<math>\scriptstyle BG+AD=2\sdot KM</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וקוי ב"ג א"ד ביחד הם כפל כ"מ |
|- | |- | ||
| | | | ||
| − | :: | + | ::<math>\scriptstyle KM=16+\frac{1}{2}</math> cubits. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכ"מ הוא י"ו אמות וחצי |
|- | |- | ||
| | | | ||
| − | :: | + | ::<math>\scriptstyle KL=12</math> cubits, because so is line AZ. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכ"ל הוא י"ב אמות כי כן הוא קו א"ז |
|- | |- | ||
| | | | ||
| − | : | + | :Therefore the area of this trapezoid is 198 cubits. |
| − | + | |style="text-align:right;"|א"כ תשבורת הנוטה הזה הוא קצ"ח אמות | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| + | !<span style=color:Green>Obtuse trapezoid</span> | ||
| | | | ||
| − | |||
|- | |- | ||
| | | | ||
| − | *If you wish to measure | + | *If you wish to measure an obtuse trapezoid, such as trapezoid ABGD, whose angle B is obtuse, AB is 13 cubits, GD is 20 cubits, AG is 6 cubits, and BD is 17 cubits. |
| − | |style="text-align:right;"|<big> | + | |style="text-align:right;"|<big>אם</big> רצית למדוד נוטה נרוח הזויות כמו נוטה אבג"ד והזוית אשר אצל ב' נרוחת והיה צלע א"ב י"ג אמות וצלע ג"ד כ' אמות וצלע א"ג ו' אמות וצלע ב"ד י"ז אמות |
|- | |- | ||
| | | | ||
| − | : | + | :Like this: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כזה |
|- | |- | ||
| | | | ||
| − | : | + | ::Subtract 6 cubits from 17 cubits; 11 cubits remain. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|תגרע הו' אמות מהי"ז אמות וישארו י"א אמות |
|- | |- | ||
| | | | ||
| − | : | + | ::Since the sides of the obtuse triangle are known - 13, 17, 20 - the height is also found, which is 12. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ובהיות צלעי המשלש נרוח הזוית ידועות י"ג וי"ז וכ' ימצא גם העמוד שהוא י"ב |
|- | |- | ||
| | | | ||
| − | :: | + | ::Add 17 to 6; it is 23. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|תחבר הי"ז עם הו' הם כ"ג |
|- | |- | ||
| | | | ||
| − | :: | + | ::Its half is 11 and a half. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ומחצית זה הם י"א וחצי |
|- | |- | ||
| | | | ||
| − | :: | + | ::Multiply it by 12; it is 138 and this is the area of this trapezoid. |
| − | ::<math>\scriptstyle{\color{blue}{\left | + | ::<math>\scriptstyle{\color{blue}{\left[\frac{1}{2}\sdot\left(17+6\right)\right]\sdot12=\left(\frac{1}{2}\sdot23\right)\sdot12=\left(11+\frac{1}{2}\right)\sdot12=138}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|תכפלם על י"ב יהיו קל"ח והוא תשבורת הנוטה הזה |
|- | |- | ||
| | | | ||
| − | * | + | *The proof of this measuring: |
| − | |style="text-align:right;"|<big> | + | |style="text-align:right;"|<big>והמופת</big> על זאת המדידה |
|- | |- | ||
| | | | ||
| − | : | + | :*I draw height AH. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אוציא עמוד א"ה |
|- | |- | ||
| | | | ||
| − | : | + | :*I draw line AZ parallel to line GD. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואוציא קו א"ז נכחי לקו ג"ד |
|- | |- | ||
| | | | ||
| − | :* | + | ::Therefore: |
| − | |style="text-align:right;"| | + | :*AZ is 20 cubits. |
| + | |style="text-align:right;"|יהיה א"כ קו א"ז כ' אמות | ||
|- | |- | ||
| | | | ||
| − | : | + | :*ZD is 6 cubits. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וקו ז"ד ו' אמות |
|- | |- | ||
| | | | ||
| − | : | + | :*So, the remaining BZ is 11 cubits. |
| − | + | |style="text-align:right;"|ואם כן קו ב"ז הנשאר יהיה י"א אמות | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | ::Since <math>\scriptstyle\triangle ABZ</math> is obtuse, line AH is 12 cubits. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ולכן לפי שמשלש אב"ז הוא נרוח הזוית יהיה קו א"ה י"ב אמות |
|- | |- | ||
| | | | ||
| − | : | + | :As explained above, <math>\scriptstyle\left(BD+AG\right)\sdot AH</math> is double the trapezoid ABGD. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכן לפי מה שהתבאר למעלה השטח אשר יהיה מב"ד א"ג ביחד ומא"ה הוא כפל נוטה אבג"ד |
|- | |- | ||
| | | | ||
| − | : | + | :Hence, the area of this trapezoid is 138 cubits. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אם כן תשבורת הנוטה הזה הוא קל"ח אמות |
|- | |- | ||
| + | !<span style=color:Green>Scalene trapezoid</span> | ||
| | | | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | *If you wish to measure a trapezoid, such that none of its sides are parallel, angle G is right, and each of its sides is known: AB is 13 cubits, BG is 10 cubits, GD is 20 cubits, and DA is 17 cubits. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<big>ואם</big> רצית למדוד נוטה אבג"ד ולא יהיה צלע מצלעותיה נכוחי והזוית אשר אצל ג' נצבת ויהיה כל צלע מצלעותיה ידוע אם צלע א"ב י"ג אמות ואם ב"ג י' אמות ואם ג"ד כ' אמות ‫<ref>49v</ref>ואם ד"א י"ז אמות כזה |
|- | |- | ||
| | | | ||
| − | :: | + | :Do as follows: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|תעשה כך |
|- | |- | ||
| | | | ||
| − | :: | + | ::Multiply the 10 cubits by the 20 cubits; it is 200. |
| − | + | |style="text-align:right;"|תכפול הי' אמות על הכ' אמות ויהיו ר‫' | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | ::Take its half; it is 100. | |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\frac{1}{2}\sdot\left(10\sdot20\right)=\frac{1}{2}\sdot200=100}}</math> |
| + | |style="text-align:right;"|וקח מחציתם והוא ק‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | ::Multiply the 10 by itself; it is 100. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{10^2=100}}</math> |
| + | |style="text-align:right;"|ועוד תכפול הי' על עצמם ויהיו ק‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | ::And the 20 by itself; it is 400. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{20^2=400}}</math> |
| + | |style="text-align:right;"|והכ' על עצמם ויהיו ת‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Sum them; it is 500. |
| + | ::<math>\scriptstyle{\color{blue}{10^2+20^2=100+400=500}}</math> | ||
| + | |style="text-align:right;"|וחברם ויהיו ת"ק | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::The 13 by itself; it is 169. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{13^2=169}}</math> |
| + | |style="text-align:right;"|והי"ג על עצמם ויהיו קס"ט | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::Add it to 500; it is 669. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{10^2+20^2+13^2=500+169=669}}</math> |
| + | |style="text-align:right;"|חברם עם הת"ק ויהיו תרס"ט | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::Subtract from it the square of 17; 380 remains. |
| − | ::<math>\scriptstyle{\color{blue}{ | + | ::<math>\scriptstyle{\color{blue}{\left(10^2+20^2+13^2\right)-17^2=669-17^2=380}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|גרע מהם מרובע י"ז וישארו ש"פ |
|- | |- | ||
| | | | ||
| − | + | ::Take its half; it is 190. | |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\frac{1}{2}\sdot\left[\left(10^2+20^2+13^2\right)-17^2\right]=\frac{1}{2}\sdot380=190}}</math> |
| + | |style="text-align:right;"|קח חציים והוא ק"צ | ||
|- | |- | ||
| | | | ||
| − | : | + | ::Multiply it by itself; it is 36100. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\left[\frac{1}{2}\sdot\left[\left(10^2+20^2+13^2\right)-17^2\right]\right]^2=190^2=36100}}</math> |
| + | |style="text-align:right;"|כפלם על עצמם ויהיו ל"ו אלפי' וק‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | ::Divide it by 500; the quotient is 72 and one-fifth. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\frac{\left[\frac{1}{2}\sdot\left[\left(10^2+20^2+13^2\right)-17^2\right]\right]^2}{10^2+20^2}=\frac{36100}{500}=72+\frac{1}{5}}}</math> |
| + | |style="text-align:right;"|חלקם על ת'[ק'] ויהיה החלק ע"ב וחמישית | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Subtract it from 169; the remainder is 96, a half, one-fifth, and one-tenth. |
| + | |style="text-align:right;"|תגרעם מן קס"ט ישארו צ"ו וחצי וחמישית ועשירית | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | ::<math>\scriptstyle{\color{blue}{13^2-\frac{\left[\frac{1}{2}\sdot\left[\left(10^2+20^2+13^2\right)-17^2\right]\right]^2}{10^2+20^2}=169-\left(72+\frac{1}{5}\right)=96+\frac{1}{2}+\frac{1}{5}+\frac{1}{10}}}</math> | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::Multiply it by 500; it is 48400. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כפלם על ת"ק ויהיו מ"ח אלף ות‫' |
| + | |- | ||
| + | |colspan=2| | ||
| + | ::<math>\scriptstyle{\color{blue}{\left[13^2-\frac{\left[\frac{1}{2}\sdot\left[\left(10^2+20^2+13^2\right)-17^2\right]\right]^2}{10^2+20^2}\right]\sdot\left(10^2+20^2\right)=\left(96+\frac{1}{2}+\frac{1}{5}+\frac{1}{10}\right)\sdot500=48400}}</math> | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::Extract its root; it is 22[0]. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|קח גדרם ויהיו רכ"א |
|- | |- | ||
| | | | ||
| − | :: | + | ::Take its half; it is 110 and this is the area of <math>\scriptstyle\triangle ABD</math>. |
| − | ::<math>\scriptstyle{\color{blue}{\frac{1}{2}\sdot\left | + | |style="text-align:right;"|וקח מחציתם ויהיה ק"י והוא תשבורת משלש אב"ד |
| − | + | |- | |
| + | |colspan=2| | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{1}{2}\sdot\sqrt{\left[13^2-\frac{\left[\frac{1}{2}\sdot\left[\left(10^2+20^2+13^2\right)-17^2\right]\right]^2}{10^2+20^2}\right]\sdot\left(10^2+20^2\right)}=\frac{1}{2}\sdot\sqrt{48400}=\frac{1}{2}\sdot220=110}}</math> | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::But, the area of <math>\scriptstyle\triangle BGD</math> is 100 cubits. |
| + | |style="text-align:right;"|אבל גם תשבורת משולש בג"ד ק' אמות | ||
|- | |- | ||
| | | | ||
| − | + | :Therefore, the area of trapezoid ABGD is 210 cubits. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אם כן תשבורת נוטה אבג"ד ר"י אמות |
|- | |- | ||
| | | | ||
| − | + | *The proof of this measuring: | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<big>המופת</big> על המדידה הזאת |
|- | |- | ||
| | | | ||
| − | : | + | :We draw line BD. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|נגיע קו ב"ד |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :I place height AH on it. |
| + | |style="text-align:right;"|ואעמיד עליה עמוד קו א"ה | ||
|- | |- | ||
| | | | ||
| − | : | + | :Since each of the sides BG and GD are known, and <math>\scriptstyle\measuredangle G=90^\circ</math>, then <math>\scriptstyle\triangle BGD</math> is known. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ולפי שכל אחד מצלעי ב"ג ג"ד הם ידועות וזוית שאצל ג' נצבת אם כן משלש בג"ד יהיה ידוע |
|- | |- | ||
| | | | ||
| − | : | + | :So, the square of BD is also known and it is 500 cubits. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{BD^2=500}}</math> |
| + | |style="text-align:right;"|ויהיה גם מרובע ב"ד ידוע והוא ת"ק אמות | ||
|- | |- | ||
| | | | ||
| − | : | + | :But the square of AB is also known. |
| − | + | |style="text-align:right;"|אבל גם מרובע א"ב ידוע | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | :Therefore, the squares of AB and BD are known and [their sum] is greater than the square of AD. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|א"כ מרובעי א"ב ב"ד ידועים והם יותר ממרובע א"ד |
|- | |- | ||
| | | | ||
| − | : | + | :So, <math>\scriptstyle\measuredangle ABD</math> is acute angle. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אם כן זוית אב"ד תהיה חדה |
|- | |- | ||
| | | | ||
| − | : | + | :The [sum of] the squares of AB and BD exceeds the square of AD by double the rectangle encompassed by lines DB and BH. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואם כן מרובעי א"ב ב"ד גדולים ממרובע א"ד בכפל השטח הנצב הזויות אשר יקיפו בו קוי [‫ד"ב ב"ה |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :So, double the rectangle encompassed by lines DB and BH is known, therefore once the rectangle encompassed by lines DB and BH is also know and it is the root of [the product of] the square of BD by the square of BH. |
| + | |style="text-align:right;"|אם כן כפל השטח הנצב הזוית אשר יקיפו בו ד"ב ב"ד ידוע לכן גם השטח הנצב הזוית אשר יקיפו בו קוי ד"ב]‫<ref>om.</ref> א' ב"ה פעם אחת ידוע והוא גדר מרובע ב"ד על מרובע ב"ה | ||
|- | |- | ||
| | | | ||
| − | : | + | :Hence, [the product of] the square of DB by the square of BH is known. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אם כן גם מרובע ד"ב על מרובע ב"ה ידוע |
|- | |- | ||
| | | | ||
| − | :: | + | :The square of BD is known. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ומרובע ב"ד ידוע |
| + | |- | ||
| + | | | ||
| + | :So, the square of BH is known. | ||
| + | |style="text-align:right;"|א"כ גם מרובע ב"ה ידוע | ||
|- | |- | ||
| | | | ||
| − | : | + | :But so is [the product of] the square of HA by the square of BD and its root is the [rectangle] encompassed by lines BD and DH. |
| − | + | |style="text-align:right;"|אבל גם מרובע ה"א על מרובע ב"ד וגדרו הוא השטח אשר יקיפו ב"ד [ד"ה]‫<ref>P1031 א"ה</ref> | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Therefore, the [rectangle] encompassed by BD and DH is known and it is double <math>\scriptstyle\triangle ABD</math>. |
| + | |style="text-align:right;"|א"כ גם השטח אשר יקיפו בו ב"ד ד"ה ידוע והוא כפל משלש אב"ד | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :So, <math>\scriptstyle\triangle ABD</math> is known. |
| + | |style="text-align:right;"|אם כן גם משלש אב"ד ידוע | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :But <math>\scriptstyle\triangle BGD</math> is also known. |
| + | |style="text-align:right;"|אבל גם משלש בג"ד ידוע | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Hence the whole quadrangle ABGD is known. |
| + | |style="text-align:right;"|ולכן כל בעלת ד' צלעות אבג"ד ידועה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *Since the height drawn from point A to line GD is known, I bring a proof of it: |
| + | |style="text-align:right;"|והיות גם העמוד היוצא מנקדת א' על קו [ג"ד]‫<ref>P1031 ב"ד</ref> ידוע הנה אני מביא עליו המופת | ||
|- | |- | ||
| | | | ||
| − | : | + | :Let the trapezoid on it be ABGD, each of its sides are known and <math>\scriptstyle\measuredangle BGD=90^\circ</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|יהיה נוטה עליו אבג"ד וכל אחת מצלעותיו תהיה ידועה וזוית בג"ד נצבת |
|- | |- | ||
| | | | ||
| − | : | + | :Since the height drawn from point A to line GD is known, I draw also height AZ on line GD, height BC on line AZ, and height AH on line BD. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והיות העמוד היוצא מנקדת א' על קו ג"ד ידוע אוציא אם על קו ג"ד עמוד א"ז ועל קו א"ז עמוד ב"ח ועל קו ב"ד עמוד א"ה |
|- | |- | ||
| | | | ||
| − | : | + | :It is clear that line BD is known and so is height AH on it, since BA and AD are known. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והוא מבואר כי קו ב"ד ידוע וא"ה העמוד אשר עליה לפי שגם ב"א א"ד הם ידועים |
|- | |- | ||
| | | | ||
| − | : | + | :Since <math>\scriptstyle\measuredangle GBD=\measuredangle BTA</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ולפי שזוית גב"ד שוה לזוית בט"א |
|- | |- | ||
| | | | ||
| − | : | + | :But also <math>\scriptstyle\measuredangle BGD=90^\circ=\measuredangle AHT</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אבל גם זוית בג"ד הנצבת שוה לזוית אה"ט הנצבת |
|- | |- | ||
| | | | ||
| − | : | + | :Then, <math>\scriptstyle DG:GB=AH:HT</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אם כן [כיחס ד"ג אל ג"ב כן]‫<ref>P1031 om.</ref> יחס א"ה אל ה"ט |
|- | |- | ||
| | | | ||
| − | :: | + | :<math>\scriptstyle DG:GB</math> is known, therefore <math>\scriptstyle AH:HT</math> is also known. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ויחס ג"ד אל ג"ב ידוע א"כ גם יחס א"ה אל ה"ט ידוע |
|- | |- | ||
| | | | ||
| − | : | + | :AH is known, therefore HT is also known. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וא"ה ידוע אם כן גם ה"ט ידוע |
|- | |- | ||
| | | | ||
| − | : | + | :They encompass a right angle, therefore AT is also known, |
| − | + | |style="text-align:right;"|והם מקיפים בזוית נצבת אם כן גם א"ט ידוע | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | : | + | :Since BH and HT each are known, then the rectangle encompassed by BTH is known and it is equal to the [rectangle] encompassed by ATC. |
| − | |style="text-align:right;"|אם כן | + | |style="text-align:right;"|ולפי שכל אחת מב"ה ה"ט ידוע אם כן גם השטח נצב הזויות ידוע אשר יקיפו בו בט"ה ויהיה שוה לשטח אשר יקיפו בו אט"ח |
|- | |- | ||
| | | | ||
| − | : | + | :<math>\scriptstyle\measuredangle H=90^\circ=\measuredangle C</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והזוית אשר אצל כל אחת מה' ח' נצבת |
|- | |- | ||
| | | | ||
| − | : | + | :So, CT is also known, and therefore AC is also known. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אם כן גם ח"ט ידוע ולכן יהיה גם א"ח ידוע |
|- | |- | ||
| | | | ||
| − | : | + | :But <math>\scriptstyle CZ=BG</math>, therefore the whole AZ is known. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אבל גם ח"ז שוה לב"ג אם כן גם א"ז כולו יהיה ידוע |
|- | |- | ||
| | | | ||
:The procedure is as follows: | :The procedure is as follows: | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ויהיה אופן המעשה כן |
|- | |- | ||
| | | | ||
| − | : | + | :*If AB is 13 cubits, BG is 10 cubits, GD is 20 cubits, and DA is 17 cubits; and we wish to find the area mentioned. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אם צלע א"ב יהיה י"ג אמו' ואם צלע ב"ג י' אמות ואם צלע ג"ד כ' אמות ואם צלע ד"א י"ז אמו' ‫<ref>50r</ref>ונרצה למצוא התשבורת הנז‫' |
|- | |- | ||
| | | | ||
| − | :: | + | ::*If the square of height AH is 96, a half, a fifth, and one part of 15; |
| − | |style="text-align:right;"| | + | :::<math>\scriptstyle{\color{blue}{AH^2=96+\frac{1}{2}+\frac{1}{5}+\frac{1}{15}}}</math> |
| + | |style="text-align:right;"|ויהיה אם עמוד א"ה בכח צ"ו וחצי [וחמישית וחלק]‫<ref>P1031: וחמש יתחלק א'</ref> מט"ו | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::*And if the square of BH is 72 and a fifth; |
| − | |style="text-align:right;"| | + | :::<math>\scriptstyle{\color{blue}{BH^2=72+\frac{1}{5}}}</math> |
| + | |style="text-align:right;"|ואם ב"ה יהיה בכח ע"ב [וחמישית]‫<ref>P1031: וחמש</ref> | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::*And if the square of BD is 500; |
| − | |style="text-align:right;"| | + | :::<math>\scriptstyle{\color{blue}{BD^2=500}}</math> |
| + | |style="text-align:right;"|ואם ב"ד יהיה בכח ת"ק | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::Since GD is 20 cubits and GB is ten cubits, then their squares are 400 cubits and 100 cubits, do as follows: |
| + | |style="text-align:right;"|ולפי שאם צלע ג"ד היא כ' אמות ואם ג"ב היא עשר אמות א"כ מרובעם יהיה ת' אמות וק' אמות ועשה כך | ||
| + | |- | ||
| | | | ||
| + | ::Examine as the ratio of 400 to 100, [to how much] is the ratio to 96, a quarter and a fifth? | ||
| + | |style="text-align:right;"|ראה כיחס ת' אל הק' מה היחס אל צ"ו ורביעית וחמישית | ||
|- | |- | ||
| | | | ||
| − | + | ::You find that it is 24 and a fifth; and so is the square of HT. | |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{400:100=\left(96+\frac{1}{4}+\frac{1}{5}\right):\left(24+\frac{1}{5}\right)}}</math> |
| + | |style="text-align:right;"|ותמצא שהוא אל כ"ד וחמישית וכן מרבע ה"ט | ||
|- | |- | ||
| | | | ||
| − | : | + | ::Multiply the 72 by 24 and a fifth, extract the root of the product and multiply it by two; the result is double the rectangle encompassed by BH and HT. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכפל הע"ב על כ"ד וחמישית וקח גדר ההוה וכפלהו בשנים וההוה הוא ככפל השטח הנצב הזוית אשר יקיפו בו ב"ה [ה"ט‫]‫<ref>P1031 om.</ref> |
|- | |- | ||
| | | | ||
| − | : | + | ::We sum up the squares of BH and HT, i.e. 72 and a fifth with 24 and a fifth. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ונוסיף מרובע ב"ה [ה"ט]‫<ref>P1031 ב"ט</ref> כלומר מרובע ע"ב וחמישית וכ"ד וחמישית מחוברים |
|- | |- | ||
| | | | ||
| − | : | + | ::We know the square of BT, which is 180. |
| − | :<math>\scriptstyle\left( | + | ::<math>\scriptstyle{\color{blue}{BT^2=180}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ונדע ב"ט בכח שהוא ק"פ |
| + | |- | ||
| + | | | ||
| + | ::Sum up 96, a half, a fifth, and a tenth, with 24 and a fifth; the result is 124. | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(96+\frac{1}{2}+\frac{1}{5}+\frac{1}{10}\right)+\left(24+\frac{1}{5}\right)=124}}</math> | ||
| + | |style="text-align:right;"|וחבר הצ"ו וחצי וחמישית ועשירית והכ"ד וחמישיתו ויעלה קכ"ד | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::Multiply 180 by 24 and a fifth; it is the square 4356. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{180\sdot\left(24+\frac{1}{5}\right)=4356}}</math> |
| + | |style="text-align:right;"|וכפל הק"ף על הכ"ד וחמישית יהיה בכח ד' אלפים שנ"ו | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::Divide it by 121; it is 36. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\frac{4356}{121}=36}}</math> |
| + | |style="text-align:right;"|וחלקם על הקכ"א יהיה ל"ו | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::We subtract the [root of] 36 from the [root of] 121; the [root of] 25 remains, which is 5 in length. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\sqrt{121}-\sqrt{36}=\sqrt{25}=5}}</math> |
| + | |style="text-align:right;"|ונגרע מקכ"א בכח ל"ו בכח וישארו כ"ה בכח שהם ה' באורך | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::Add to it the whole size of BG, which is 10 cubits; the result is the size of the height. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{5+10=15}}</math> |
| + | |style="text-align:right;"|ותוסיף כל שעור ב"ג והוא י' אמות וההוה ט"ו וזהו שעור העמוד | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::The square of HT is 24 and a fifth, the length of CT is 6, and the length of AT is 11. |
| − | |style="text-align:right;"|ואם | + | |style="text-align:right;"|ואם ה"ט בכח כ"ד וחמישית ואם ח"ט באורך ו' ואם [א"ט]‫<ref>P1031 om.</ref> באורך י"א |
|- | |- | ||
| | | | ||
| − | : | + | :*If you wish to measure trapezoid ABGD, of which <math>\scriptstyle\measuredangle G=90^\circ</math>; AB is 13 cubits, BG is 10 cubits, GD is 8 cubits, and AD is 25 cubits. You want to find its area. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<big>ואם</big> תרצה למדוד נוטה אבג"ד אשר הזוית שאצל ג' נצבת ויהיה צלע א"ב י"ג אמות וצלע ב"ג י' אמות וצלע ג"ד ח' אמות וצלע א"ד כ"ה אמות כזה ותרצה למצוא תשברתו |
|- | |- | ||
| | | | ||
| − | :: | + | ::Do as follows: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|תעשה כן |
|- | |- | ||
| | | | ||
| − | :: | + | ::Multiply the 10 by itself; it is 100. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{10^2=100}}</math> |
| + | |style="text-align:right;"|תכפול הי' על עצמם יעלו ק‫' | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::Multiply also the 8 by itself; it is 64. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{8^2=64}}</math> |
| + | |style="text-align:right;"|גם הח' על עצמם יעלו ס"ד | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle | + | ::Sum them; it is 164. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{10^2+8^2=100+64=164}}</math> |
| + | |style="text-align:right;"|תחברם יעלו קס"ד | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::Multiply also the 13 by itself; it is 169. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{13^2=169}}</math> |
| + | |style="text-align:right;"|גם תכפול הי"ג על עצמם יעלו קס"ט | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle | + | ::Sum them; it is 333. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{10^2+8^2+13^2=164+169=333}}</math> |
| + | |style="text-align:right;"|חברם עמם יעלו של"ג | ||
|- | |- | ||
| | | | ||
| − | : | + | ::Multiply also the 25 by itself; it is 625. |
| − | :<math>\scriptstyle\ | + | ::<math>\scriptstyle{\color{blue}{25^2=625}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|גם כפול הכ"ה על עצמם יעלו תרכ"ה |
|- | |- | ||
| | | | ||
| − | : | + | ::Subtract 333; 292 remains. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{25^2-\left(10^2+8^2+13^2\right)=625-333=292}}</math> |
| + | |style="text-align:right;"|גרע של"ג ישארו רצ"ב | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::Take its half; it is 146. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\frac{1}{2}\sdot\left[25^2-\left(10^2+8^2+13^2\right)\right]=\frac{1}{2}\sdot292=146}}</math> |
| + | |style="text-align:right;"|קח חציים והם קמ"ו | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::Multiply it by itself; it is 21316. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\left[\frac{1}{2}\sdot\left[25^2-\left(10^2+8^2+13^2\right)\right]\right]^2=146^2=21316}}</math> |
| + | |style="text-align:right;"|כפלם על עצמם יעלו כ"א אלף שי"ו | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::Divide it by 164; the quotient is 129 and 160 parts of 164. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\frac{\left[\frac{1}{2}\sdot\left[25^2-\left(10^2+8^2+13^2\right)\right]\right]^2}{10^2+8^2}=\frac{21316}{164}=129+\frac{160}{164}}}</math> |
| + | |style="text-align:right;"|חלקם על קס"ד יהיה החלק קכ"ט וק"ס חלקים מקס"ד באחד | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::Subtract it from 169; 39 and 4 parts of 164 remain. |
| − | + | |style="text-align:right;"|גרעם מן קס"ט ישארו ל"ט וד' חלקים מן קס"ד באחד | |
| + | |- | ||
| + | |colspan=2| | ||
| + | ::<math>\scriptstyle{\color{blue}{13^2-\frac{\left[\frac{1}{2}\sdot\left[25^2-\left(10^2+8^2+13^2\right)\right]\right]^2}{10^2+8^2}=169-\left(129+\frac{160}{164}\right)=39+\frac{4}{164}}}</math> | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::Multiply them by 164; it is 6400. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כפלם על קס"ד יעלו ו' אלפים ות‫' |
|- | |- | ||
| | | | ||
| − | :: | + | ::Its root is 80. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וגדרו פ‫' |
|- | |- | ||
| | | | ||
| − | :: | + | ::Its half is 40; so the area of <math>\scriptstyle\triangle ABD</math> is 40 cubits. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וחציים מ' ויהיה תשבורת משולש אב"ד מ' אמות |
|- | |- | ||
| + | |colspan=2| | ||
| + | ::<math>\scriptstyle{\color{blue}{\begin{align}&\scriptstyle\frac{1}{2}\sdot\sqrt{\left[13^2-\frac{\left[\frac{1}{2}\sdot\left[25^2-\left(10^2+8^2+13^2\right)\right]\right]^2}{10^2+8^2}\right]\sdot\left(10^2+8^2\right)}\\&\scriptstyle=\frac{1}{2}\sdot\sqrt{\left(39+\frac{4}{164}\right)\sdot164}=\frac{1}{2}\sdot\sqrt{6400}=\frac{1}{2}\sdot80=40\\\end{align}}}</math> | ||
| | | | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | :: | + | ::But, the area of <math>\scriptstyle\triangle BGD</math> is also 40 cubits. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אבל גם תשבורת משולש בג"ד כן מ' אמות |
|- | |- | ||
| | | | ||
| − | :: | + | ::Therefore, the area of trapezoid ABGD is 80 cubits. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אם כן תשבורת כל נוטה אבג"ד פ' אמות |
|- | |- | ||
| | | | ||
| − | : | + | *The proof of this measuring: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והמופת על המדידה הזאת |
|- | |- | ||
| | | | ||
| − | : | + | :We draw line BD. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|נגיע ב"ד |
|- | |- | ||
| | | | ||
| − | : | + | :The area of <math>\scriptstyle\triangle BGD</math> is known. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ויהיה תשבורת משולש בג"ד ידוע |
|- | |- | ||
| | | | ||
| − | :: | + | :The square of BD is 164 cubits. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{BD^2=164}}</math> |
| + | |style="text-align:right;"|ומרובע ב"ד יהיה קס"ד אמות | ||
|- | |- | ||
| | | | ||
| − | : | + | :But the square of AB is 169. |
| − | + | :<math>\scriptstyle{\color{blue}{AB^2=169}}</math> | |
| − | |style="text-align:right;"|א" | + | |style="text-align:right;"|אבל גם מרובע א"ב קס"ט אמות |
|- | |- | ||
| | | | ||
| − | : | + | :So, [the sum of] the squares of AB and BD is 333, which is less than the square of AD. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{AB^2+BD^2=333<AD^2}}</math> |
| + | |style="text-align:right;"|א"כ מרובע א"ב ב"ד [הם]‫<ref>P1031: ככפל השטח הנצב הזויות שהם</ref> של"ג אמות והם פחותים ממרובע א"ד | ||
|- | |- | ||
| | | | ||
| − | :Hence, | + | :Hence, <math>\scriptstyle\measuredangle ABD</math> is obtuse. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אם כן זוית אב"ד נרוחת |
|- | |- | ||
| | | | ||
| − | : | + | :I draw height AH on DB, by extending it to H. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואוציא עמוד א"ה על ד"ב בהוציאי אותו עד ה‫' |
| − | |- | + | |- |
| − | | | + | | |
| − | |style="text-align:right;"| | + | :So, the square of AD exceeds [the sum of] the squares of AB and BD by double the rectangle encompassed by lines BD and BH. |
| + | :<math>\scriptstyle{\color{blue}{AD^2-\left(AB^2+BD^2\right)=2\sdot BD\sdot BH}}</math> | ||
| + | |style="text-align:right;"|א"כ מרובע א"ד יותר גדול ממרובעי א"ב ב"ד ככפל השטח הנצב הזויו' אשר יקיפו בו [ב"ד ב"ה | ||
|- | |- | ||
| | | | ||
| − | : | + | :Hence, double the rectangle encompassed by lines BD and BH is known. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אם כן כפל השטח הנצב הזויות אשר יקיפו בו]‫<ref>P1031 om.</ref> קוי [ב"ד]‫<ref>P1031 om.</ref> ב"ה ידוע |
|- | |- | ||
| | | | ||
| − | : | + | :Therefore, the rectangle encompassed by lines BD and BH is also known and it is the root of [the product of] the square of BD by the square of BH. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{BD\sdot BH=\sqrt{BD^2\sdot BH^2}}}</math> |
| + | |style="text-align:right;"|לכן גם השטח הנצב הזויות אשר יקיפו בו ב"ד ב"ה יהיה ידוע ויהיה גדר מרובע ב"ד על [מרובע]‫<ref>P1031 om.</ref> ב"ה | ||
|- | |- | ||
| | | | ||
| − | : | + | :So, [the product of] the square of BD by the square of BH is known. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|א"כ מרובע ב"ד על מרובע ב"ה ידוע |
|- | |- | ||
| | | | ||
| − | : | + | :The square of BD [is known], therefore the square of HB that remains is also known. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אבל גם מרובע ב"ד [אם כן]‫<ref>P1031 om.</ref> גם מרובע ה"ב הנשאר ידוע |
|- | |- | ||
| | | | ||
| − | : | + | :<math>\scriptstyle\measuredangle H=90^\circ</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והזוית אשר אצל ה' נצבת |
|- | |- | ||
| | | | ||
| − | : | + | :So the square of AH is known. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|א"כ גם מרובע א"ה ידוע |
|- | |- | ||
| | | | ||
| − | : | + | :Therefore, [the product of] the square of AH by the square of BD is known. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|לכן גם מרובע א"ה על מרובע ב"ד ידוע |
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :Its root is the rectangle encompassed by AH and BD, so the rectangle encompassed by AH and BD is also known. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ויהיה גדרו השטח הנצב הזויות אשר יקיפו בו א"ה ב"ד אם כן גם שטח הנצב הזוית אשר יקיפו בו א"ה ב"ד ידוע |
|- | |- | ||
| | | | ||
| − | : | + | :Its half is <math>\scriptstyle\triangle ABD</math>, which is 40 cubits as we noted. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וחציו הוא משלש אב"ד שהוא מ' אמות כמו שזכרנו |
|- | |- | ||
| | | | ||
| − | : | + | :So, <math>\scriptstyle\triangle ABD</math> is known, therefore the whole trapezoid ABGD is also known. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|‫<ref>50v</ref>אם כן גם משלש אב"ד ידוע לכך גם כל בעל ד' צלעות אבג"ד יהיה ידוע |
|- | |- | ||
| | | | ||
| − | + | ||
| − | |style="text-align:right;"| | + | ==== Chapter Five on Knowing the Round Shapes ==== |
| + | |||
| + | |style="width:45%; text-align:right;"|<big>הפרק החמישי</big> בידיעת התמונות העגולות | ||
|- | |- | ||
| − | | | + | |Know that the circle is a line, within which there is a point, such that all the lines drawn from it to the circumference are equal. |
| − | + | |style="text-align:right;"|דע שהעגולה היא קו אחד בתוכה נקדה שכל הקוי' היוצאים ממנה אל המקיף שוים | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| − | | | + | |This chapter includes the measurement of the whole circle that we have defined, as well as the measurement of the segments of the circle. |
| − | : | + | |style="text-align:right;"|והנה בזה השער יכנסו מדידת העגולה השלימה אשר גדרנוה ומדידת חתיכות העגולה |
| − | | | ||
|- | |- | ||
| − | | | + | |The segments of the circle are of three types: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וחתיכות העגולה ג' מינים |
|- | |- | ||
| | | | ||
| − | + | *The first is a semicircle. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|הא' הוא חצי העגולה |
|- | |- | ||
| | | | ||
| − | + | *The second is greater than a semicircle. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|השני יותר מחציה |
|- | |- | ||
| | | | ||
| − | : | + | *The third is less than a semicircle. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|השלישי פחות מחציה |
| + | |- | ||
| + | |We begin with the measuring of whole circle, then we discuss the measuring of its segments. | ||
| + | |style="text-align:right;"|ונחל במדידת העגולה השלימה אחר נדבר במדידת חתיכותיה | ||
|- | |- | ||
| + | !<span style=color:Green>Circle</span> | ||
| | | | ||
| − | :: | + | |- |
| − | |style="text-align:right;"| | + | |We say that the area of the circle is [obtained] by that you multiply half of its diameter by half of its perimeter. |
| + | |style="text-align:right;"|ונאמר כי תשבורת העגולה היא בשתכה את מחצית קטרה במחצית הקו המקיף אותה | ||
| + | |- | ||
| + | |The ratio of its perimeter, i.e. the line surrounding the circle, to its diameter is as the ratio of 22 to 7. | ||
| + | |style="text-align:right;"|והנה ערך הקו המקיף אותה ר"ל את העגולה מקטרה כערך כ"ב אל הז‫' | ||
| + | |- | ||
| + | |We find that the ratio of the perimeter is 3⅐ according to the opinion of the geometricians that are experts in this science. | ||
| + | |style="text-align:right;"|נמצא שהקו המקיף הוא בערך ג' ושביעית וזה לפי דעת חכמי המדות המדקדקים בחכמה הזאת | ||
| + | |- | ||
| + | |Ptolemy relied all his calculations in the Almagest on the calculation that the diameter is a third of the perimeter, because he divided the circle into 360 degrees and the diameter into 120 degrees, and although there is no ratio between the diameter that is a straight line and the perimeter that is a circular line, the ratio exists in a number not in the magnitude. | ||
| + | |style="text-align:right;"|אמנם {{#annot:Ptolemy|509|DEBp}}בטלמיוס{{#annotend:DEBp}} עשה כל חשבונותיו בספר המג'יסטי על חשבון שיהיה הקטר שלישית הקו המקיף כי חלק את העגולה על ש"ס מעלות והקטר על ק"כ מעלות ואעפ"י שאין יחס בין הקטר שהוא קו ישר לקו המקיף שהוא קו עגול אבל היחס יפול במספר לא בשעור | ||
|- | |- | ||
| | | | ||
| − | : | + | *If you wish to find the area of the circle from the perimeter alone: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<big>ואם</big> רצית למצוא תשבורת העגולה מהמקיף לבד |
|- | |- | ||
| | | | ||
| − | : | + | :Take the square of the perimeter by multiplying it by itself, multiply the product by 7, then take one part of 88 from the result and it is the area of the circle. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|תקח מרובע הקו המקיף וזה בשתכה אותו על עצמו וההוה תכה אותם על ז' וההוה קח מהם חלק א' מפ"ח והוא תשבורת העגולה |
|- | |- | ||
| | | | ||
| − | :: | + | :*Example: we multiply 22 by itself; it is 484. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|המשל בזה הכינו כ"ב על עצמם והם תפ"ד |
|- | |- | ||
| | | | ||
| − | :: | + | ::We multiply it 7 times; it it is 3388. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כפלנום ז' פעמים והם ג' אלפים ושפ"ח |
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle | + | ::We take from it one part of 88; it is 38½ and this is the area of the circle. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\left(22^2\sdot7\right)\sdot\frac{1}{88}=\left(484\sdot7\right)\sdot\frac{1}{88}=3388\sdot\frac{1}{88}=38+\frac{1}{2}}}</math> |
| + | |style="text-align:right;"|לקחנו מהם חלק מפ"ח והם ל"ח וחצי והוא תשבורת העגולה | ||
|- | |- | ||
| | | | ||
| − | :: | + | *Another example: if you wish to find the area of the circle from the perimeter alone: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|דמיון אחר אם רצית למצוא תשבורת העגולה מהמקיף לבד |
|- | |- | ||
| | | | ||
| − | : | + | :Add to the perimeter its half and its quarter and the result is the area of the circle. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|תוסיף על המקיף חציו ורביעיתו וההוה הוא תשבורת העגולה |
|- | |- | ||
| | | | ||
| − | * | + | :*Example: we add to the perimeter, which is 22 cubits, its half and its quarter that are 16½; the result is 38½ and this is the area of the circle. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{22+\left(\frac{1}{2}\sdot22\right)+\left(\frac{1}{4}\sdot22\right)=22+\left(16+\frac{1}{2}\right)=38+\frac{1}{2}}}</math> |
| + | |style="text-align:right;"|המשל בזה הוספנו על הקו המקיף שהוא כ"ב אמות חציו ורביעיתו שהם י"ו אמות וחצי ההוה ל"ח וחצי והם תשבורת העגולה | ||
|- | |- | ||
| | | | ||
| − | + | *If you wish to find the area of the circle from the diameter alone, without looking at the perimeter: | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<big>ואם</big> רצית תשבורת העגולה מהקטר לבד מבלתי שתביט אל הקו המקיף |
|- | |- | ||
| | | | ||
| − | : | + | :Multiply the square of the diameter by 11 and divide the product by 14; the result is the area of the circle and this is according to the calculation of the geometricians. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כפול מרובע הקטר על י"א וחלק העולה על י"ד והעולה הוא תשבורת העגולה וזה על חשבון חכמי המדות |
|- | |- | ||
| | | | ||
| − | : | + | :Ptolemy said elsewhere that the ratio of the perimeter to the whole diameter is 3 and 8 parts of sixty. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ובטלמיוס אמר במקום אחר כי ערך הקו המקיף אל כל האלכסון והוא ג' וח' חלקי' מששים באחד |
|- | |- | ||
| | | | ||
| − | : | + | :However, the difference between this calculation and the calculation of the geometricians is only a negligible thing that does not affect the calculation, therefore, we based our calculation on the opinion of the geometricians. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואין הפרש בין זה החשבון ובין חשבון חכמי המדות רק דבר מועט שאינו מזיק בחשבון לכן סמכנו את חשבוננו על דעת חכמי המדות |
|- | |- | ||
| | | | ||
| − | + | *Also, if you wish to find the area of the circle from the diameter alone: | |
| − | |style="text-align:right;"|אם | + | |style="text-align:right;"|עוד אם רצית למצוא תשבורת העגולה מהקטר לבד |
|- | |- | ||
| | | | ||
| − | : | + | :Take the diameter, multiply is by the perimeter, then take from the product it quarter and this is the area of the circle. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|תקח הקטר וכפלהו על הקו המקיף וקח מההוה רביעיתו והוא תשבורת העגולה |
|- | |- | ||
| | | | ||
| − | :: | + | :*Example: the diameter is 7 and the perimeter is 22. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|המשל בזה הקטר ז' והקו המקיף כ"ב |
|- | |- | ||
| | | | ||
| − | :: | + | ::We multiply them by each other; the result is 154. Its quarter is 38 and a half and this is the area of the circle. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\frac{1}{4}\sdot\left(7\sdot22\right)=\frac{1}{4}\sdot154=38+\frac{1}{2}}}</math> |
| + | |style="text-align:right;"|כפלנו זה על זה וההוה קנ"ד ורביעיתו ל"ח וחצי והוא תשבורת העגולה | ||
| + | |- | ||
| + | |We have already explained the method of measuring the area of the circle. | ||
| + | |style="text-align:right;"|הנה כבר בארנו דרך תשבורת מדידת העגולה | ||
|- | |- | ||
| + | !<span style=color:Green>Segments of the circle</span> | ||
| | | | ||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |Now we start to explain the method of measuring its segments. |
| − | : | + | |style="text-align:right;"|‫<ref>51r</ref><big>ומעתה</big> נחל לבאר דרך מדידת חתיכותיה |
| − | |style="text-align:right;"| | + | |- |
| + | |We say that the segment of the circle is surrounded by a segment of the perimeter and by a straight line called a chord that is drawn from the end of the perimeter to its other end. | ||
| + | |style="text-align:right;"|ונאמר כי [חתכת]‫<ref>P1031: מקצת</ref> העגולה היא מקפת מן קצת הקו המקיף את העגולה ומקו ישר יקרא המיתר והוא המשוך מקצה הקו המקיף אותה עד קצהו האחר | ||
| + | |- | ||
| + | |When a line is drawn from half the chord to the perimeter at right angle it is called versine. | ||
| + | |style="text-align:right;"|וכאשר יצא ממחצית המיתר קו על זוית נצבת אל המקיף יקרא החץ | ||
| + | |- | ||
| + | |Examine each segment: | ||
| + | |style="text-align:right;"|ועיין אל כל חתיכה | ||
|- | |- | ||
| | | | ||
| − | + | *If the versine is as half the chord, know that the segment is a semicircle. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואם החצי יהיה כמחצית המיתר דע שהחתיכה היא חצי עגולה |
|- | |- | ||
| | | | ||
| − | + | *If it is shorter than half the chord, know that the segment is less than a semicircle. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואם יהיה קצר ממחצית היתר דע שהחתיכה היא פחותה מחצי העגולה |
|- | |- | ||
| | | | ||
| − | + | *If it is longer than half the chord, the segment is greater than a semicircle. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואם הוא יותר ארוך ממחצית היתר החתיכה היא גדולה ממחצית העגולה |
|- | |- | ||
| + | !<span style=color:Green>Semicircle</span> | ||
| | | | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | + | *When the segment is a semicircle and you wish to know its area: | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכאשר היתה החתיכה חצי עגולה ורצית לדעת תשברתה |
|- | |- | ||
| | | | ||
| − | : | + | :Multiply half the chord, which is the diameter, by half the circumference and the result is the area of the semicircle. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|תכפול חצי היתר שהוא אלכסון החצי עם חצי הקו המקיף אותה וההוה הוא תשבורת מחצית העגולה |
|- | |- | ||
| | | | ||
| − | : | + | :For, half the circumference of the segment is a quarter of the perimeter of the whole circle. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כי מחצית הקו המקיף העגולה שהיא החתיכה הוא רביע הקו המקיף כל העגולה השלימה |
|- | |- | ||
| | | | ||
| − | : | + | :When you multiply half the diameter by half [the perimeter of] the circle, you find the area of the whole circle. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכאשר הכית מחצית הקטר [במחצית]‫<ref>P1031: ברביע</ref> העגולה תמצא תשבורת כל העגולה |
|- | |- | ||
| | | | ||
| − | : | + | :When you multiply half the diameter by a quarter of [the perimeter of] the circle, you find the area of the semicircle. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכאשר תכה מחצית הקטר ברביע העגולה תמצא תשבורת חצי העגולה |
|- | |- | ||
| | | | ||
| − | :: | + | ::Because, for every number you multiply its half by its whole, the result is half the product of its whole by its whole. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כי כל חשבון שתכה חציו בכולו יהיה ההוה מחצית הכאת כלו [בכלו]‫<ref>P1031 om.</ref> |
|- | |- | ||
| | | | ||
| − | :: | + | ::If you multiply its half by its half, the result is a quarter of the product of its whole by its whole. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואם תכה חציו בחציו ההוה רביע הכאת כלו בכולו |
|- | |- | ||
| | | | ||
| − | :: | + | :*Example: a whole circle whose perimeter is 22 cubits. We know that its diameter is 7 cubits. We wish to find its area. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|המשל בזה עגולה שלימה אשר הקו המקיף אותה כ"ב אמות ידענו שקטרה ז' אמות רצינו למצוא תשברתה |
|- | |- | ||
| | | | ||
| − | :: | + | ::We multiply half of the diameter, which is 3 and a half, by half the perimeter, which is 11 cubits; the result is 38 and a half. We know from this that the area of the circle is 38 cubits and a half. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\left(\frac{1}{2}\sdot7\right)\sdot\left(\frac{1}{2}\sdot22\right)=\left(3+\frac{1}{2}\right)\sdot11=38+\frac{1}{2}}}</math> |
| + | |style="text-align:right;"|הכינו מחצית הקטר שהוא ג' וחצי על מחצית הקו המקיף שהוא י"א אמות וההוה ל"ח וחצי ידענו מזה שתשבורת העגולה ל"ח וחצי אמות | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::We wish to know the area of half this circle: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|רצינו לדעת תשבורת מחצית העגולה הזאת |
|- | |- | ||
| | | | ||
| − | :: | + | ::We take half of its circumference, which is 5 and a half. We multiply it by half the diameter, which is 3 and a half; the result is 19 and a quarter and this is the area of the semicircle. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\left(\frac{1}{2}\sdot11\right)\sdot\left(\frac{1}{2}\sdot7\right)=\left(5+\frac{1}{2}\right)\sdot\left(3+\frac{1}{2}\right)=19+\frac{1}{4}}}</math> |
| + | |style="text-align:right;"|לקחנו מחצית הקו המקיף אותה שהוא ה' וחצי והכינו אותם עם מחצית הקטר שהוא ג' וחצי וההוה י"ט ורביע והוא תשבורת חצי העגולה | ||
|- | |- | ||
| | | | ||
| − | :: | + | :*Example: a semicircle whose diameter is 14 cubits and the versine is 7 cubits. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|המשל בזה חצי עגולה שיהיה קטרה י"ד אמות והחץ ז' אמות |
|- | |- | ||
| | | | ||
| − | :: | + | ::Take the square of the diameter, which is 196, multiply it by 11; the result is 2156. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|תקח מרובע הקטר והוא קצ"ו אמות ותכפלהו בי"א ההוה ב' אלפים קנ"ו |
|- | |- | ||
| | | | ||
| − | :::<math>\scriptstyle | + | ::Take one part of 28, which is 77 cubits, and this is the area of the semicircle. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\frac{1}{28}\sdot\left(14^2\sdot11\right)=\frac{1}{28}\sdot\left(196\sdot11\right)=\frac{1}{28}\sdot2156=77}}</math> |
| + | |style="text-align:right;"|קח החלק האחד מכ"ח שהם ע"ז אמות והם תשבורת חצי העגולה | ||
|- | |- | ||
| | | | ||
| − | + | *If you wish to find its area using the versine, do as follows: | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<big>ואם</big> רצית למצוא את תשבורתה מהחץ תעשה כך |
|- | |- | ||
| | | | ||
| − | : | + | :Take the square of the versine, multiply it by 11, then take its seventh and this is the area of the semicircle. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|תקח מרובע החץ ותכפלהו על י"א ותקח שביעיתו והוא תשבורת חצי העגולה |
|- | |- | ||
| | | | ||
| − | :: | + | :*Example: take the square of the versine, which is 7 and its square is 49, multiply it by 11; the result is 539 and its seventh is 77 and this is the area of the semicircle. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\frac{1}{7}\sdot\left(7^2\sdot11\right)=\frac{1}{7}\sdot\left(49\sdot11\right)=\frac{1}{7}\sdot539=77}}</math> |
| + | |style="text-align:right;"|המשל בזה קח מרובע החץ שהוא ז' ומרובעו מ"ט כפלהו על י"א ההוה תקל"ט ושביעיתו ע"ז והוא תשבורת חצי העגולה | ||
|- | |- | ||
| | | | ||
| − | : | + | :*Another semicircle, whose diameter is 7 cubits, its versine is half the diameter, which is 3½, and the perimeter is 11 cubits. You wish to know its area. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|עוד חצי עגולה אשר קטרה ז' אמות וחצה חצי הקטר שהוא ג' וחצי והקו המקיף י"א אמות ותרצה לדעת תשברתה |
|- | |- | ||
| | | | ||
| − | :: | + | ::Do as follows: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|תעשה כך |
|- | |- | ||
| | | | ||
| − | :: | + | ::Multiply the 7 cubits of the diameter by the 11 cubits of the perimeter; the result is 77 cubits; take its quarter, which is 19¼ cubits and this is the area of the semicircle. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\frac{1}{4}\sdot\left(7\sdot11\right)=\frac{1}{4}\sdot77=19+\frac{1}{4}}}</math> |
| + | |style="text-align:right;"|תכפול הז' אמות של הקטר עם הי"א אמות של הקו המקיף וההוה ע"ז אמות קח רביעיתם והם י"ט אמות ורביע והם תשבורת חצי העגולה | ||
|- | |- | ||
| | | | ||
| − | : | + | :*In another way, do as follows: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ובאופן אחר תעשה כך |
|- | |- | ||
| | | | ||
| − | + | ::Take the square of the diameter, which is 7 cubits and its square is 49, multiply it by 11; the result is 539. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|תקח מרובע הקטר שהוא ז' אמות ומרובעו מ"ט כפלם על י"א וההוה תקל"ט |
|- | |- | ||
| | | | ||
| − | : | + | ::Take one part of 28; its is 19¼ cubits and this is the area. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\frac{1}{28}\sdot\left(7^2\sdot11\right)=\frac{1}{28}\sdot\left(49\sdot11\right)=\frac{1}{28}\sdot539=19+\frac{1}{4}}}</math> |
| + | |style="text-align:right;"|קח חלק א' מכ"ח והוא י"ט אמות ורביע וזהו התשבורת | ||
|- | |- | ||
| + | !<span style=color:Green>Segment that is less than a semicircle</span> | ||
| | | | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | *We wish to find the area of a segment that is less than a semicircle: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|‫<ref>51v</ref><big>רצינו</big> למצוא תשבורת חתיכת עגולה פחות מחציה |
|- | |- | ||
| | | | ||
| − | : | + | :For this it is necessary to find the diameter of the whole circle, from which this segment is generated. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ובזה הוא הכרח למצוא קטר כל העגולה אשר זאת החתיכה ממנה |
|- | |- | ||
| | | | ||
| − | :: | + | :We do as follows: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וככה נעשה |
|- | |- | ||
| | | | ||
| − | : | + | :If has already been clarified in Euclid's Book of Elements [III.35] that when two chords in any circle cut each other the product of a part of the chord by the other [part] is as the product of a part of the other chord by its other [part]. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כבר התבאר בספר היסודות לאקלידס כי כל עגולה יפלו בה שני מיתרים ויחתכו זה את זה יהיה [כפל]‫<ref>P1931 om.</ref> חלק המיתר בחברו האחר ככפל חלק המיתר השני בחברו |
|- | |- | ||
| | | | ||
| − | : | + | :When we want to find the diameter of the circle, from which this segment is generated, we draw it so it cuts the chord at right angle, so it cuts it in the middle as explained in Euclid's Book of Elements [III.3]. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכאשר רצינו למצוא אלכסון העגולה אשר זו החתיכה נחצבת ממנו נעבירהו חותך המיתר על זויות נצבות ויחתכהו באמצע כפי מה שהתבאר בספר היסודות לאקלידס |
|- | |- | ||
| | | | ||
| − | : | + | :We multiply half the chord by its half and the product should be as the product of the versine of this segment by the rest of the diameter. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ונכפול חצי המיתר על מחציתו וההוה ממנו ראוי שיהיה כמו ההוה מחץ החתיכה הזאת על שארית האלכסון |
|- | |- | ||
| | | | ||
| − | : | + | :When we know the measure of the length of the versine, we multiply it by a number, such that the product is as the product of half the chord by its half, and [the sum of] the number, by which we multiply it, with the versine is the measure of the diameter. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכאשר ידענו כמות אורך החץ נכפלהו על מספר שיהיה ההוה מכפלו כמו ההוה ממחצי' המיתר על מחציתו והמספר אשר כפלנו אותו עליו יחד עם החץ יהיה שעור אלכסון |
|- | |- | ||
| | | | ||
| − | :: | + | :*Example: AB is a segment that is less than a semicircle; its chord is 8 cubits; its versine, which is GD, is 2 cubits and it cuts the chord at right angle. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|המשל בזה יהיה חתיכת עגולה פחות מחציה חתיכת א"ב ויהיה מיתרה ח' אמות וחצה ב' אמות והוא ג"ד חותך על זויות נצבות את המיתר |
|- | |- | ||
| | | | ||
| − | :: | + | ::It necessarily cuts it in half. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ובהכרח שיחתוך אותו אל חצאים |
|- | |- | ||
| | | | ||
| − | :: | + | ::When we extend it to the perimeter of the circle in order to complete it, it passes through the center of the circle. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכשנוציאנו עד מקיף העגולה כאשר נשלים אותה יעבור על מרכז העגולה |
|- | |- | ||
| | | | ||
| − | :: | + | ::It cuts the chord to 4 and 4, so when we multiply half of the chord by its half, the result is 16. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\left(\frac{1}{2}\sdot8\right)\sdot\left(\frac{1}{2}\sdot8\right)=4\sdot4=16}}</math> |
| + | |style="text-align:right;"|והנה חתך המיתר על ד' ד' אם כן כשנעריך חצי המיתר על חציו ההוה י"ו | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::We have already assumed that the versine is 2. We multiply it by a number, such that the result will by 16; this number is exactly 8. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{2\sdot8=16}}</math> |
| + | |style="text-align:right;"|וכבר הנחנו החץ ב' נעריך אותו על מספר שיהיה ההוה י"ו ואין מספר כזה זולתי הח‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | ::We know that the missing part from the versine until the completion of the diameter is 8. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וידענו שהחסר מן החץ עד תשלום האלכסון הם ח‫' |
|- | |- | ||
| | | | ||
| − | + | ::We sum it with the versine; it is 10 and this is the length of the diameter. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|חברנום עם החץ ביחד והיו י' והוא אורך כל האלכסון |
|- | |- | ||
| | | | ||
| − | : | + | ::We make this shape and complete the circle: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ונעשה [כזאת הצורה]‫<ref>P1031: חצו בה</ref> ונשלים העגולה |
|- | |- | ||
| | | | ||
| − | :: | + | ::We say that we draw two lines that are half the diameter from point C, which is the center of the circle, to point A and point B. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ונאמר שנוציא שני קוים מנקדת ח' שהוא חצי האלכסון ומרכז העגולה אל נקדת א' ונקדת ב‫' |
|- | |- | ||
| | | | ||
| − | :: | + | ::We multiply half the diameter, which is CG, by half the arch surrounding the segment, which is arch AG; the result is the area of the triangle, whose two sides are CA and CB, and whose base is arch ABG. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ונכה מחצית הקטר שהוא ח"ג במחצית הקשת המקיף את החתיכה והוא קשת א"ג וההוה הוא תשבורת המשולש אשר שתי צלעותיו ח"א ח"ב ותושבתו קשת אב"ג |
|- | |- | ||
| | | | ||
| − | :: | + | ::Then, we multiply half the chord by the height of the triangle, which is line CD; the result is the area of the triangle of straight lines. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אחר כך נכה מחצית המיתר בעמוד המשולש הוא קו [ח"ד]<ref>P1031 א"ד</ref> וההוה הוא תשבורת המשולש ישר הקוים |
|- | |- | ||
| | | | ||
| − | :: | + | ::We subtract this area from the area of the triangle, whose base is arch ABG; the remainder is the required area of the segment. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|נגרע התשבורת [הזאת]<ref>P1031 om.</ref> מתשבורת המשולש אשר תושבותיו קשת אב"ג ואשר ישאר הוא תשבורת החתיכה הדרושה |
|- | |- | ||
| | | | ||
| − | : | + | *When we want to know this measure in another way, without having to extract the whole diameter: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<big>וכאשר</big> רצינו לדעת זאת המדידה באופן אחר מבלתי שנצטרך להוציא כל אלכסון העגולה |
|- | |- | ||
| | | | ||
| − | : | + | :We sum up the chord and the versine, take their half and multiply it by the versine. We multiply also half the chord by itself and take one part of fourteen from the product of half the chord by itself. We add it to the product of half the chord and the versine by the versine and this is the area. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|נחבר המיתר והחץ ונקח חציים ונכה אותם עם החץ גם נכה חצי המיתר על עצמו ונקח מהכאת חצי המיתר על עצמו חלק א' מן ארבעה עשר ונוסיף אותם על הכאת חצי המיתר והחצי עם החץ והוא התשבורת |
|- | |- | ||
| | | | ||
| − | :: | + | :*Such as: a chord, which is 12, with a versine, which is 4; they are 16 and their half is 8. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כגון מיתר י"ב עם חץ ד' שהם י"ו וחציים ח‫' |
| + | |- | ||
| + | | | ||
| + | ::We multiply it by 4; it is 32. | ||
| + | ::<math>\scriptstyle{\color{blue}{\left[\frac{1}{2}\sdot\left(12+4\right)\right]\sdot4=\left(\frac{1}{2}\sdot16\right)\sdot4=8\sdot4=32}}</math> | ||
| + | |style="text-align:right;"|נכה בד' הם ל"ב | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle\ | + | ::Half the chord by itself is 36. One part of 14 of it is 2 cubits, a half and one part of 14. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\frac{1}{14}\sdot\left(\frac{1}{2}\sdot12\right)^2=\frac{1}{14}\sdot36=2+\frac{1}{2}+\frac{1}{14}}}</math> |
| + | |style="text-align:right;"|וחצי המיתר על עצמו הם ל"ו חלקו מי"ד ב' אמות וחצי וחלק מי"ד | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::We add them to 32 cubits; the area of the segment is 34 cubits, a half and one part of 14. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|נוסיפם ‫<ref>52r</ref>על הל"ב אמות יהיה תשבורת החתיכה ל"ד אמות וחצי וחלק א' מי"ד |
| + | |- | ||
| + | |colspan=2| | ||
| + | ::<math>\scriptstyle{\color{blue}{\left[\left[\frac{1}{2}\sdot\left(12+4\right)\right]\sdot4\right]+\left[\frac{1}{14}\sdot\left(\frac{1}{2}\sdot12\right)^2\right]=32+\left(2+\frac{1}{2}+\frac{1}{14}\right)=34+\frac{1}{2}+\frac{1}{14}}}</math> | ||
|- | |- | ||
| + | !<span style=color:Green>Segment that is greater than a semicircle</span> | ||
| | | | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | *We wish to find the area of a segment that is greater than a semicircle: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<big>נרצה</big> למצוא תשבורת חתיכת העגולה אשר היא גדולה מחציה |
|- | |- | ||
| | | | ||
| − | : | + | :*Let this segment be arch ABG with chord AG. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והחתיכה הזאת היא קשת אב"ג עם מיתר א"ג |
|- | |- | ||
| | | | ||
| − | : | + | :You can know the area when you find the diameter of the circle that this segment is its segment: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ותוכל לדעת התשבורת כשתמצא קטר העגולה אשר זאת החתיכה היא חתיכה ממנה |
|- | |- | ||
| | | | ||
| − | : | + | :Multiply half the diameter by half the arc, then add to it the area of the triangle, whose base is the chord and whose top is the center of the circle; the result is the area of the segment. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ותהיה כופל חצי הקטר בחצי הקשת ותהיה מוסיף עליו תשבורת המשולש אשר תושבתו המיתר וראשו מרכז העגולה וההוה הוא תשבורת החתיכה |
|- | |- | ||
| | | | ||
| − | :: | + | :We shall delve into the knowledge of the diameter: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ונעמוד על ידיעת האלכסון כן |
|- | |- | ||
| | | | ||
| − | : | + | :We take the square of half the chord, divide it by the versine, add the quotient to the versine; the result is the diameter of the circle, from which this segment is cut. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|נקח מרובע חצי המיתר ונחלקהו על החץ ונוסיף העולה על החץ וההוה הוא אלכסון העגולה אשר זאת החתיכה נחתכה ממנה |
|- | |- | ||
| | | | ||
| − | : | + | :*Example: arch ABG is a segment of a circle; the chord is AG, whose length is 12 cubits; and the versine is BD, whose length is 8 cubits. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|המשל בזה יהיה חתיכת העגולה קשת אב"ג והמיתר א"ג וארכו י"ב אמות והחץ ב"ד וארכו ח' אמות |
|- | |- | ||
| | | | ||
| − | + | ::We take the square of half the chord, which is 36, divide it by the versine, which is 8; the quotient is 4 cubits and a half. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|לקחנו מרובע חצי היתר שהוא ל"ו וחלקנוהו על החץ שהוא ח' ועלה החלק ד' אמות וחצי |
|- | |- | ||
| | | | ||
| − | : | + | ::We add it to the versine; it is 12 cubits and a half, so we know that the diameter of is 12 cubits and a half. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\frac{\left(\frac{1}{2}\sdot12\right)^2}{8}+8=\frac{36}{8}+8=\left(4+\frac{1}{2}\right)+8=12+\frac{1}{2}}}</math> |
| + | |style="text-align:right;"|הוספנום על החץ והם י"ב אמות וחצי וידענו שהאלכסון הוא י"ב אמות וחצי | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::We wish to find the area: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|רצינו למצוא את התשבורת |
|- | |- | ||
| | | | ||
| − | :: | + | ::We take half the diameter, which is 6 cubits and a quarter, multiply it by half the arc, and keep the result. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ולקחנו חצי הקטר שהוא ו' אמות ורביע וכפלנו אותו על חצי הקשת וההוה שמרנוהו |
|- | |- | ||
| | | | ||
| − | :: | + | ::Then, we find the area of the triangle, whose base is AG, its height is HD and its two sides are AH and GH. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אחר כך מצאנו תשבורת המשולש [שתושבתו]‫<ref>P1031: תשברתו</ref> א"ג ועמודו ה"ד ושתי צלעותיו הם א"ה ג"ה |
|- | |- | ||
| | | | ||
| − | :: | + | ::We find it as follows: we know that the length of each of its two sides is as half the diameter, therefore, the square of the side is as [the sum of] the square of the height and the square of half the chord, because it is a base of a right-angled [triangle]. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ומצאנוהו כן ידענו שאורך כל א' מצלעותיו השתים הוא כארך חצי הקטר ולכן יהיה מרובע הצלע כמרובע העמוד ומרובע מחצי' המיתר כי הוא תושבת לזוית הנצבת |
|- | |- | ||
| | | | ||
| − | :: | + | ::Since the square of half the chord is 36, we subtract it from the square of the side, which is 39 cubits and one part of 16; 3 cubits and one part of 16 remain and they are the square of the length of the height. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\left(39+\frac{1}{16}\right)-\left(\frac{1}{2}\sdot12\right)^2=\left(39+\frac{1}{16}\right)-36=3+\frac{1}{16}}}</math> |
| + | |style="text-align:right;"|ואחרי שמרובע מחצית המיתר ל"ו נגרעהו ממרובע הצלע שהוא ל"ט אמות וחלק א' מי"ו נשארו ג' אמות וחלק א' מי"ו והם מרובע ארך העמוד | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::We multiply the height by half the base, which is 6 cubits; the result is 18 cubits, a third, one part of 30, and one part of 120, and this is the area of <math>\scriptstyle \triangle AHG</math>. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\left(3+\frac{1}{16}\right)\sdot\left(\frac{1}{2}\sdot12\right)=\left(3+\frac{1}{16}\right)\sdot6=18+\frac{1}{3}+\frac{1}{30}+\frac{1}{120}}}</math> |
| + | |style="text-align:right;"|כפלנו העמוד עם מחצי' התושבת שהם ו' אמות וההוה הם י"ח אמות ושלישית וחלק מל' גם אחד מק"כ והוא תשבורת משלש אה"ג | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::We add it to the reserved and the result is the area of the segment mentioned. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|הוספנו על השמור וההוה הוא תשבורת החתיכה הנזכרת |
|- | |- | ||
| | | | ||
| − | + | *If we want to find this area, without having to extract the diameter, we do as follows: | |
| − | |style="text-align:right;"|ואם | + | |style="text-align:right;"|<big>ואם</big> רצינו למצוא התשבורת הזאת מבלי שנצטרך להוציא האלכסון נעשה כך |
|- | |- | ||
| | | | ||
| − | : | + | :We multiply the chord by the versine, multiply the product by 11, then take one part of 14 of the result and this is the area of this segment. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|נכה המיתר עם החץ וההוה נכה אותו עם י"א ומן ההוה נקח חלק א' מי"ד והוא תשבורת החתיכה הזאת |
|- | |- | ||
| | | | ||
| − | * | + | :*Example: arch ABG is a segment that is greater than a semicircle, its chord is AG, whose length is 24 cubits, and the versine is 16 cubits. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|המשל בזה חתיכת עגולה גדולה מחציה היא קשת אב"ג ומיתרה א"ג וארכו כ"ד אמות והחץ י"ו אמות |
|- | |- | ||
| | | | ||
| − | : | + | ::We multiply the versine by the chord; the result is 384 cubits. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|הכינו החץ עם המיתר ההוה שפ"ד אמות |
|- | |- | ||
| | | | ||
| − | :: | + | ::We multiply 384 by 11; the result is 4 thousand and 224. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|הכינו שפ"ד עם י"א ההוה ד' אלפים ורכ"ד |
|- | |- | ||
| | | | ||
| − | :: | + | ::We take one part of 14 of it; the result is 301, a half, a seventh, and one part of 14, and this is the area of the segment mentioned. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\frac{1}{14}\sdot11\sdot\left(24\sdot16\right)=\frac{1}{14}\sdot\left(11\sdot384\right)=\frac{1}{14}\sdot4224=301+\frac{1}{2}+\frac{1}{7}+\frac{1}{14}}}</math> |
| + | |style="text-align:right;"|לקחנו מהם חלק א' מהי"ד וההוה ש"א אמות וחצי ושביעית וחלק מי"ד והוא תשבורת החתיכה הנזכרת | ||
|- | |- | ||
| + | !<span style=color:Green>Finding the arc</span> | ||
| | | | ||
| − | : | + | |- |
| − | |style="text-align:right;"| | + | |Said Mordecai: since for these measurements we need to find the size of the arc surrounding the segments of the circle and this is explained in the tables of the chords and arcs, because by knowing the chord we know the arc and by knowing the arc we know the chord, as Ptolemy explained in the first book of the Almagest, and writing tables is not the way of our book, I saw fit to show you a way to know the arcs without having to use these tables. |
| + | |style="text-align:right;"|<big>אמר</big> מרדכי בעבור שאלה המדידות אנו צריכים למצוא כמות הקשת המקפת בחתיכו' העגולה וזה מתבאר בלוחות המיתרים והקשתות כי בידיעת המיתר נדע הקשת גם בידיעת הקשת נדע המיתר כמו שביאר זה בטלמיוס במאמר א' מספר האלמג'יסטי ‫<ref>52v</ref>מאג'יסטי ואין מדרך חבורנו זה לכתוב בו לוחות ראיתי להמציא לך דרך לדעת הקשתות מבלי שתצטרך אל הלוחות ההם | ||
|- | |- | ||
| | | | ||
| − | : | + | *I say that if the segment is less than a semicircle and you wish to know the size of the arc surrounding it, do as follows: |
| − | |style="text-align:right;"|אם | + | |style="text-align:right;"|ואומר אם חתכת העגולה תהיה פחות מחציה ותרצה לדעת כמות הקשת המקיף אותה תעשה כך |
|- | |- | ||
| | | | ||
| − | : | + | :Always sum the chord with the versine, subtract a quarter [of the sum], take a quarter of the remainder and add it [to the remainder]; the result is the size of the arc surrounding the segment. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|לעולם תחבר המיתר על החץ ותגרע מהם רביעיתם וקח מהנשארים רביעיתם והוסף עליהם וההוה הוא כמות הקשת המקיף את החתיכה |
|- | |- | ||
| | | | ||
| − | :: | + | :*Example: AB is a segment that is less than a semicircle, its chord is 40 cubits and its versine is 10 cubits. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|המשל בזה תהיה חתיכת עגולה פחות מחציה חתיכת א"ב ומיתרה מ' אמות וחצה י' אמות |
|- | |- | ||
| | | | ||
| − | :: | + | ::We sum up the chord and the versine; the result is 50 cubits. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{40+10=50}}</math> |
| + | |style="text-align:right;"|חברנו המיתר עם החץ ההוה נ' אמות | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle | + | ::We subtract from it 12 cubits and a half, which are its quarter; 37 cubits and a half remain. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{50-\left(\frac{1}{4}\sdot50\right)=50-\left(12+\frac{1}{2}\right)=37+\frac{1}{2}}}</math> |
| + | |style="text-align:right;"|גרענו אלו י"ב אמות וחצי שהם רביעיתם נשארו ל"ז אמות וחצי | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle | + | ::We take 9 cubits, a quarter and an eighth, which are a quarter of the 37 cubits and a half that remain. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\frac{1}{4}\sdot\left(37+\frac{1}{2}\right)=9+\frac{1}{4}+\frac{1}{8}}}</math> |
| + | |style="text-align:right;"|לקחנו ט' אמות ורביע ושמינית שהם רביע הל"ז אמות וחצי אשר נשארו | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::We add them to them; the result is 46 cubits, 3-quarters and an eighth, and this is the size of the circumference of the segment. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והוספנו אותם עליהם ההוה מ"ו אמות וג' רביעיות ושמינית והוא כמות הקו המקיף את החתיכה |
| + | |- | ||
| + | |colspan=2| | ||
| + | ::<math>\scriptstyle{\color{blue}{\left[\left(40+10\right)-\frac{1}{4}\sdot\left(40+10\right)\right]+\frac{1}{4}\sdot\left[\left(40+10\right)-\frac{1}{4}\sdot\left(40+10\right)\right]=\left(37+\frac{1}{2}\right)+\left(9+\frac{1}{4}+\frac{1}{8}\right)=46+\frac{3}{4}+\frac{1}{8}}}</math> | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::We subtract a quarter and add a quarter, since the versine is a quarter of the chord. |
| − | + | |style="text-align:right;"|אמנם גרענו רביע והוספנו רביע בעבור שהחץ הוא רביעית חלק מהמיתר | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| + | !<span style=color:Green>Shapes with curved sides</span> | ||
| | | | ||
| − | + | |- | |
| − | |style="text-align:right;"| | + | |Having explained all of these, it is now possible to measure all shapes with a curved side. |
| + | |style="text-align:right;"|ואחר שבארנו [כל אלה]‫<ref>P1031 om.</ref> מעתה אתה יכול למדוד כל הצורות שיש להם צלע עקום | ||
|- | |- | ||
| | | | ||
| − | + | *Such as triangle ABG, whose two sides are straight and its base is a rounded arch, which is arch BG. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כגון משלש אב"ג אשר שתי צלעותיו ישרים ותושבתה קשת עגול והוא קשת ב"ג |
|- | |- | ||
| | | | ||
| − | : | + | :Measure the triangle by itself, by drawing a straight line from B to G. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|שתמדוד המשלש בפני עצמו וזה בשתמשוך קו ישר מב' עד ג‫' |
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | + | :Draw the height on line BG. | |
| − | = | + | |style="text-align:right;"|ותוציא העמוד על קו ב"ג |
| + | |- | ||
| | | | ||
| + | :Multiply it by half the base, as in the measurement of the triangles. | ||
| + | |style="text-align:right;"|ותכהו במחצית התושבת כמו במדידת המשלשים | ||
|- | |- | ||
| | | | ||
| − | + | :We find also the arc, by finding the circle, from which it is generated, as we explained in measuring the segments that are less than a semicircle or greater than a semicircle. | |
| − | + | |style="text-align:right;"|עוד נמצא את הקשת הזה במצאינו עגולה מאיזו נחצבה כמו שבארנו במדידת החתיכות שהם פחותות מחצי עגולה או עודפות על חצי עגולה | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| − | |We | + | | |
| − | |style="text-align:right;"| | + | :We find its area and add it to the area of the triangle; the sum is the area of this shape. |
| + | |style="text-align:right;"|ונמצא תשבורתה ונחבר אותה עם תשבורת המשולש והמחובר הוא תשבורת התמונה הזאת | ||
|- | |- | ||
| − | | | + | |We do so also when there are two curved sides and the other is a straight line, like this: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|גם ככה נעשה בהיות שתי הצלעות עקומו' כזה והאחרת קו ישר |
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | *Such as shape ABG: |
| + | |style="text-align:right;"|כגון תמונת אב"ג | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :We draw two straight lines from points A and B to point G. |
| + | |style="text-align:right;"|שנמשך שני קוים ישרים מנקודת א' וב' אל נקדת ג‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | :We construct an equilateral triangle and measure it according to the rule. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ונעשה משלש ישר הקוים ונמדוד אותו כמשפט |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :We also measure the two curves, each being a part of a circle, since the two sides of the triangle are chords of the two segments mentioned. |
| + | |style="text-align:right;"|עוד נמדוד שני העקומות כל אחת בהיותה חתיכת עגולה כי שתי צלעות המשולש הם מיתרים לשתי החתיכות הנזכרות | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :We sum up the three areas, i.e. the area of the triangle and the areas of each of the two segments; the result is the area of this shape. |
| + | |style="text-align:right;"|ונחבר שלשת המדידות כלומר מדידת המשולש ומדידת כל אחת משתי החתיכות וההוה הוא תשבורת תמונה הזאת | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|גם ככה | + | *Likewise if its two sides are curved and by them the shape is completed, as shape ABG: |
| + | |style="text-align:right;"|גם ככה אם היו שתי צלעותיה עקומות ובם תשלם התמונה כמו תמונת אב"ג | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :We draw a straight line from point B to point D, so it is a chord of the two segments of the circle - segment BAD and segment BGD. |
| + | |style="text-align:right;"|שנוציא קו ישר מנקדת ב' אל נקדת ד' ויהיה מיתר לשתי חתיכות של העגולה לחתיכת בא"ד ולחתיכת בג"ד | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :We measure each segment by itself, then sum up the two areas and this is the area of the shape. |
| + | |style="text-align:right;"|ונמדוד כל חתיכה בפני עצמה ונחבר שתי המדידות והם יהיו תשבורת התמונה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | |
| + | ==== Chapter Six on the Measuring of Regular Polygons ==== | ||
| + | |||
| + | |style="width:45%; text-align:right;"|<big>הפרק הששי</big> במדידת התמונות רבות הזויות | ||
| + | |- | ||
| + | |know that every polygon is divided into triangles, even the triangle itself. | ||
| + | |style="text-align:right;"|דע שכל תמונה ישרת הקוים תחלק אל משלשים ואפי' המשולש עצמו | ||
| + | |- | ||
| + | |As explained in the books of the ancients that the triangle is a root and a foundation for all the polygons, because they are formed from it and decomposed to it. | ||
| + | |style="text-align:right;"|כמו שהתבאר בספרי הקדמונים שהמשולש הוא שרש ויסוד לכל התמונות ישרות הקוים <ref>53r</ref>כי מהם התהוו ואליהם יותכו | ||
| + | |- | ||
| + | |You already know the measurement of the various triangles. | ||
| + | |style="text-align:right;"|וכבר ידעת מדידת המשולשים למיניהם | ||
| + | |- | ||
| + | |When you want to measure a polygon, draw a straight line from each of its vertices to the center of the shape, then divide it into triangles as the number of the vertices. | ||
| + | |style="text-align:right;"|<big>וכאשר</big> תרצה למדוד תמונה רבת הזויות תוציא קו ישר מכל זוית מזויותיו אל מרכז התמונה ואז תחלק למשלשי' בכמות הזויות | ||
| + | |- | ||
| + | |When you measure all the triangles and sum their values, the sum is the area of this shape. | ||
| + | |style="text-align:right;"|וכאשר תמדוד כל המשלשים ותקבץ מנינם המקובץ הוא תשבורת התמונה ההיא | ||
| + | |- | ||
| + | |If the sides of the triangles are equal, i.e. their bases, and so are their legs, so that you can draw a circle, [whose center is] the center of the shape, that touches each of its vertices, you measure one triangle alone and find its area. | ||
| + | |style="text-align:right;"|ואם צלעות המשולש שוים כלומר תושבותיהם גם השוקים שלהם וזה כשתעגל ממרכז התמונה ובמרחק זוית מזויותיה עגולה ותמשש כל הזויות אז תמדוד המשלש הא' לבד ותמצא תשברתו | ||
| + | |- | ||
| + | |Then you multiply the area mentioned by the number of the vertices and the result is the area of this shape. | ||
| + | |style="text-align:right;"|אח"כ תכה את התשבורת הנזכרת במנין הזויות וההוה הוא תשבורת התמונה | ||
| + | |- | ||
| + | |This is the rule that is given for the measurement of the polygons. | ||
| + | |style="text-align:right;"|וזהו הכלל הנתון במדידת התמונות רבות הזויות | ||
| + | |- | ||
| + | |In order to make it easier for the one who calculates, the scholars gave [other] ways to measure them, so we will write them here. | ||
| + | |style="text-align:right;"|אמנם כדי להקל על המחשב נתנו החכמים דרכים במדידתם ולכן נכתבם פה | ||
|- | |- | ||
| − | | | + | |We start by measuring the pentagon [lit. a shape that has five sides and five vertices], because we have already mentioned the triangles and the rectangles. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ונחל ממדידת תמונה בעלת ה' צלעות ובעלת ה' זויות כי המשלשים גם המרובעים כבר זכרנום |
|- | |- | ||
| − | | | + | |The shapes that we will mention are those that the circle touches their vertices [= cyclic polygons] and that are equilateral and equiangular [= regular polygons]. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואלה התמונות אשר נזכיר הם שתמשש העגולה זויותיהם ותהיינה שוה הצלעות והזויות |
|- | |- | ||
| − | | | + | |We say: |
| − | : | + | |style="text-align:right;"|ונאמר |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| + | !<span style=color:Green>Pentagon</span> | ||
| | | | ||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|אם | + | *If you wish to measure a pentagon |
| + | |style="text-align:right;"|אם רצית למדוד תמונה בעלת חמש זויות | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Do as follows: |
| + | |style="text-align:right;"|תעשה ככה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Multiply the side by itself, then multiply the product 5 times and take its third and this is the area of the mentioned shape. |
| + | |style="text-align:right;"|תכפול הצלע על עצמו וההוה כפלהו ה' פעמים וקח שלישיתו והוא תשבורת התמונה הנזכרת | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*Example: we have a pentagon ABGDH, each of its sides is 10 cubits. |
| + | |style="text-align:right;"|המשל יש לנו תמונת אבגד"ה בעלת ה' זויות וכל צלע מצלעותיה י' אמות | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::We multiply 10 by itself; the result is 100. |
| + | |style="text-align:right;"|כפלנו הי' על עצמו וההוה ק‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::We multiply it by 5; the result is 500. |
| + | |style="text-align:right;"|כפלנו זה על ה' וההוה ת"ק | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::We take its third; it is 166⅔ cubits and this is the area of the shape. |
| + | ::<math>\scriptstyle{\color{blue}{\frac{1}{3}\sdot\left(10^2\sdot5\right)=\frac{1}{3}\sdot\left(100\sdot5\right)=\frac{1}{3}\sdot500=166+\frac{2}{3}}}</math> | ||
| + | |style="text-align:right;"|לקחנו שלישיתם והם קס"ו אמות וב' שלישי אמה והם תשבורת התמונה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::If you want to find the diameter of the circumscribed circle of the shape, multiply the side, which is ten cubits, by 17; the product is 170. Divide it by 10; the result is 17 and this is the diameter of the circle. |
| + | ::<math>\scriptstyle{\color{blue}{\frac{10\sdot17}{10}=\frac{170}{10}=17}}</math> | ||
| + | |style="text-align:right;"|ואם תרצה למצוא גם קטר העגולה הממששת התמונה תכפול הצלע שהם עשרה אמות על י"ז ההוה ק"ע חלקם על י' וההוה י"ז והם קטר העגולה | ||
|- | |- | ||
| + | !<span style=color:Green>Hexagon</span> | ||
| | | | ||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|<big>ואם</big> רצית | + | *If you wish to measure a hexagon |
| + | |style="text-align:right;"|<big>ואם</big> רצית למדוד תמונת בעלת שש צלעות ושש זויות | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Do as follows: |
| + | |style="text-align:right;"|תעשה כך | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Multiply the side by itself, then multiply the product by 6 and take a third and a tenth of it and this is the area of the shape. |
| + | |style="text-align:right;"|תכפול הצלע על עצמו וההוה כפלהו על ו' ותקח מהם השלישית והעשירית והם תשבורת התמונה | ||
|- | |- | ||
| | | | ||
| − | + | :*Example: we have a [hexagon] ABGDHW, each of its sides is 30 cubits and the diameter is 60 cubits. | |
| − | + | |style="text-align:right;"|המשל יש לנו תמונת אבגדה"ו וכל צלעותיה ל' אמות והקטר ס' אמות | |
| − | |||
| − | |style="text-align:right;"| | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | ::We multiply 30 by itself; the result is 900. |
| + | |style="text-align:right;"|כפלנו הל' על עצמו וההוה תת"ק | ||
|- | |- | ||
| − | |||
| | | | ||
| + | ::We multiply it by 6; the result is 5400. | ||
| + | |style="text-align:right;"|כפלנום על ו' ההוה ה' אלפים וארבע מאות | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | ::We take its third and its tenth; it is 2340 and this is the area of the shape. |
| + | |style="text-align:right;"|לקחנו מהם השלישית והעשירית ההוה ב' אלפים ש"מ והוא תשבורת התמונה | ||
|- | |- | ||
| − | | | + | |colspan=2| |
| − | + | ::<math>\scriptstyle{\color{blue}{\left(\frac{1}{3}+\frac{1}{10}\right)\sdot\left(30^2\sdot6\right)=\left(\frac{1}{3}+\frac{1}{10}\right)\sdot\left(900\sdot6\right)=\left(\frac{1}{3}+\frac{1}{10}\right)\sdot5400=2340}}</math> | |
|- | |- | ||
| + | !<span style=color:Green>Heptagon</span> | ||
| | | | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | + | *If you wish to measure a heptagon | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<big>ואם</big> רצית למדוד תמונה בעלת שבע זויות |
|- | |- | ||
| | | | ||
| − | : | + | :Do as follows: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|תעשה כך |
|- | |- | ||
| | | | ||
| − | : | + | :Multiply the side by itself, then multiply the result by 43 and take one part of 12 of the product and the result is the area of the shape. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|תכפול הצלע על עצמו וההוה כפול אותו על מ"ג ומההוה קח חלק א' מי"ב וההוה הוא תשבורת התמונה |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*Example: we have a [heptagon] ABGDHWZ, each of its sides is 10 cubits. |
| + | |style="text-align:right;"|המשל יש לנו תמונת אבגדהו"ז וכל צלע מצלעותיה י' אמות | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::We multiply 10 by itself, then multiply the result by 43; it is 4300. |
| + | |style="text-align:right;"|כפול הי' על עצמם ההוה כפלם על מ"ג ההוה ד' אלפים ש‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | ::Take one part of 12; the result is 358⅓ and this is the area of the shape. |
| − | :<math>\scriptstyle\ | + | ::<math>\scriptstyle{\color{blue}{\frac{1}{12}\sdot\left(10^2\sdot43\right)=\frac{1}{12}\sdot4300=358+\frac{1}{3}}}</math> |
| − | |style="text-align:right;"|< | + | |style="text-align:right;"|קח חלק <ref>53v</ref>אחד מי"ב וההוה שנ"ח ושליש והם תשבורת התמונה |
|- | |- | ||
| + | !<span style=color:Green>Octagon</span> | ||
| | | | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | + | *If you wish to measure an octagon | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<big>ואם</big> רצית למדוד תמונה בעלת שמנה זויות |
|- | |- | ||
| | | | ||
| − | : | + | :Do as follows: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|תעשה כך |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Multiply the side by itself, then multiply the product by 29 and take its sixth and this is the area of the shape. |
| + | |style="text-align:right;"|תכפול הצלע על עצמו וההוה כפלהו על כ"ט וקח ששיתו והוא תשבורת התמונה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*Example: we have an [octagon] ABGDHWZC, each of its sides is 10 cubits. |
| − | |- | + | |style="text-align:right;"|המשל יש לנו תמונת אבגדהוז"ח וכל צלע מצלעותיה י' אמות |
| + | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::We multiply 10 by itself; the result is 100. |
| + | |style="text-align:right;"|כפלנו הי' על עצמם העולה ק‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::We multiply the 100 by 29; the result is 2900. |
| + | |style="text-align:right;"|כפלנו הק' על כ"ט ההוה ב' אלפים תת"ק | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|לקחנו | + | ::We take its sixth; the result is 483⅓ and this is the area of the shape. |
| + | ::<math>\scriptstyle{\color{blue}{\frac{1}{6}\sdot\left(10^2\sdot29\right)=\frac{1}{6}\sdot\left(100\sdot29\right)=\frac{1}{6}\sdot2900=483+\frac{1}{3}}}</math> | ||
| + | |style="text-align:right;"|לקחנו את ששיתו ההוה תפ"ג ושליש והוא תשבורת התמונה | ||
|- | |- | ||
| + | !<span style=color:Green>Nonagon</span> | ||
| | | | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *If you wish to measure a nonagon |
| + | |style="text-align:right;"|<big>ואם</big> רצית למדוד תמונה בעלת ט' צלעות וט' זויות | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Do as follows: |
| + | |style="text-align:right;"|תעשה כך | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Multiply the side by itself, then multiply the product by 51 and take its eighth and this is the area of the shape. |
| + | |style="text-align:right;"|תכפול הצלע על עצמו וההוה כפלהו על נ"א וקח שמיניתם והוא תשבורת התמונה | ||
|- | |- | ||
| | | | ||
| − | :* | + | :*Example: we have a [nonagon] ABGDHWZCT, each of its sides is 10 cubits. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|המשל יש לנו תמונת אבגדהוזח"ט וכל צלע מצלעותיה י' אמות |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::We multiply 10 by itself; the result is 100. |
| + | |style="text-align:right;"|כפלנו העשרה על עצמם ההוה ק‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::We multiply it by 51; the result is 5100. |
| + | |style="text-align:right;"|כפלנום על נ"א וההוה ה' אלפים ק‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | ::We take its eighth; the result is 637½ and this is the area of the shape. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\frac{1}{8}\sdot\left(10^2\sdot51\right)=\frac{1}{8}\sdot\left(100\sdot51\right)=\frac{1}{8}\sdot5100=637+\frac{1}{2}}}</math> |
| + | |style="text-align:right;"|לקחנו שמיניתם והם תרל"ז אמות וחצי וככה תשבורת התמונה | ||
|- | |- | ||
| + | !<span style=color:Green>Decagon</span> | ||
| | | | ||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *If you wish to measure a decagon |
| + | |style="text-align:right;"|<big>ואם</big> רצית למדוד תמונה בעלת י' צלעות וי' זויות | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Do as follows: |
| + | |style="text-align:right;"|תעשה כך | ||
|- | |- | ||
| | | | ||
| − | : | + | :Multiply the side by itself, then take the product and multiply it by 15 and take its half and this is the area of the shape. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|תכפול הצלע על עצמו וההוה קח אותו וכפלהו על ט"ו וקח חצי ההוה והוא תשבורת התמונה |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*Example: we have a [decagon] ABGDHWZCTI, each of its sides is 10 cubits. |
| + | |style="text-align:right;"|והמשל בזה יש לנו תמונת אבגדהוזחט"י וכל צלע מצלעותיה י' אמות | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::We multiply 10 by itself; it is 100. |
| + | |style="text-align:right;"|כפלנו הי' על עצמם והיו ק‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::We multiply it by 15; the result is 1500. |
| + | |style="text-align:right;"|כפלנום על ט"ו ההוה אלף ת"ק | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle\ | + | ::Its half is 750 and this is the area of the shape. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\frac{1}{2}\sdot\left(10^2\sdot15\right)=\frac{1}{2}\sdot\left(100\sdot15\right)=\frac{1}{2}\sdot1500=750}}</math> |
| + | |style="text-align:right;"|וחציים שבע מאות וחמשי' וככה תשבורת התמונה | ||
|- | |- | ||
| | | | ||
| − | : | + | ::In another way: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ובפנים האחרים גם כן |
|- | |- | ||
| | | | ||
| − | : | + | ::We multiply 10 by itself; the result is 100. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כפלנו הי' על עצמם וההוה ק‫' |
|- | |- | ||
| | | | ||
| − | : | + | ::We multiply it by 38; the result is 3800. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כפלנום על ל"ח וההוה ג' אלפים ות"ת |
| − | |- | + | |- |
| | | | ||
| − | :<math>\scriptstyle\ | + | ::We take its fifth; it is 760; this is the area of the shape and this number is more accurate. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\frac{1}{5}\sdot\left(10^2\sdot38\right)=\frac{1}{5}\sdot\left(100\sdot38\right)=\frac{1}{5}\sdot3800=760}}</math> |
| + | |style="text-align:right;"|לקחנו חמישיתו והם תש"ס והוא תשבורת התמונה וזה החשבון יותר מדוקדק | ||
|- | |- | ||
| + | !<span style=color:Green>Hendecagon</span> | ||
| | | | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | + | *If you wish to measure a hendecagon | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<big>ואם</big> רצית למדוד תמונה בעלת י"א זויות |
|- | |- | ||
| | | | ||
| − | : | + | :Do as follows: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|עשה כך |
|- | |- | ||
| | | | ||
| − | : | + | :Multiply the side by itself, then multiply the product by 66 and take from the result its seventh and this is the area of the shape. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|תכפול הצלע על עצמו וההוה כפלהו על ס"ו ומן ההוה קח שביעיתו והוא תשבורת התמונה |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*Example: we have a [hendecagon] ABGDHWZCTIU, each of its sides is 10 cubits. |
| + | |style="text-align:right;"|המשל יש לנו תמונת אבגדהוזחטי י"א וכל צלע מצלעותיה י' אמות | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::We multiply the side by itself; the result is 100. |
| + | |style="text-align:right;"|כפלנו הצלע על עצמו ההוה ק‫' | ||
|- | |- | ||
| | | | ||
| − | + | ::We multiply it by 66; the result is 6600. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כפלנום על ס"ו והיו ו' אלפים ת"ר |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::We take its seventh; it is 943 and this is the area of the shape. |
| + | ::<math>\scriptstyle{\color{blue}{\frac{1}{7}\sdot\left(10^2\sdot66\right)=\frac{1}{7}\sdot\left(100\sdot66\right)=\frac{1}{7}\sdot6600\approx943}}</math> | ||
| + | |style="text-align:right;"|לקחנו שביעיתם והם תתקמ"ג וככה תשבורת התמונה | ||
|- | |- | ||
| + | !<span style=color:Green>Dodecagon</span> | ||
| | | | ||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | + | *If you wish to measure a dodecagon | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<big>ואם</big> רצית למדוד תמונה בעלת י"ב זויות |
|- | |- | ||
| | | | ||
| − | : | + | :Do as follows: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|תעשה כך |
|- | |- | ||
| | | | ||
| − | : | + | :Multiply the side by itself, then multiply the product by 45 and take from the result its quarter and this is the area of the shape. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|תכפול הצלע על <ref>54r</ref>עצמו וההוה כפלהו על מ"ה ומההוה קח רביעיתם וככה תשבורת התמונה |
|- | |- | ||
| | | | ||
| − | :* | + | :*Example: we have a [dodecagon] ABGDHWZCTIUX, each of its sides is 10 cubits. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|המשל יש לנו תמונת א'ב'ג'ד'ה'ו'ז'ח'ט'י' י"א י"ב וכל צלע מצלעותיה י' אמות |
|- | |- | ||
| | | | ||
| − | : | + | ::We multiply the side by itself; it is 100. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כפלנו הצלע על עצמו היו ק‫' |
|- | |- | ||
| | | | ||
| − | : | + | ::We multiply it by 45; the result is 4500. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כפלנום על מ"ה וההוה ד' אלפים ת"ק |
|- | |- | ||
| | | | ||
| − | : | + | ::We take its quarter; it is 1125 and this is its area. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\frac{1}{4}\sdot\left(10^2\sdot45\right)=\frac{1}{4}\sdot\left(100\sdot45\right)=\frac{1}{4}\sdot4500=1125}}</math> |
| + | |style="text-align:right;"|לקחנו רביעיתם והם אלף קכ"ה וככה תשברתם | ||
|- | |- | ||
| − | | | + | |The other polygons that are not equilateral and equiangular are measured by measuring the triangles into which they are divided. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ושאר התמונות רבות הזויות אשר אינן שוות הצלעות והזויות נמדדות במדידת המשולשים אשר עליהן תחלקנה |
| + | |- | ||
| + | |Said Mordecai: I have taught you the measurement of the polygons from the pentagon to the dodecagon easily and without effort. | ||
| + | |style="text-align:right;"|<big>אמר מרדכי</big> הנה הודעתיך מדידת התמונות רבות הזויות מן המחומש עד בעל י"ב זויות בקלות ובלי יגיעה | ||
| + | |- | ||
| + | |I did not bring proof to every shape so as not to confuse the student, I only brought the procedure. | ||
| + | |style="text-align:right;"|אמנם לא הבאתי המופת על כל תמונה ותמונה כדי שלא אבלבל התלמיד אבל הבאתי אופן המעשה [בלבד | ||
|- | |- | ||
| − | | | + | |Now, I will bring a proof for the measurement of each shape and start with the pentagon, as I started the procedure. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ועתה אביא המופת בעבור כל מדידת תמונה ותמונה ואחל מהמחומש]‫<ref>P1031: וטרם שאביא המופת על כל תמונה ותמונה ועתה אחל המופת מן המחומש</ref> כמו שהחלותי באופן המעשה |
|- | |- | ||
| − | | | + | |Before I begin with the proof I will mention two triangles that will help to us in what we want to explain: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|‫[וטרם שאחל להביא המופת אקדים להזכיר]‫<ref>P1031: ואקים תחלה</ref> שני משולשים להיותם לעזר במה שנרצה לבאר |
|- | |- | ||
| + | !<span style=color:Green>Equilateral triangle</span> | ||
| | | | ||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :We say that <math>\scriptstyle\triangle ABG</math> is equilateral, each of its sides is 10 cubits. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ונאמר משולש אב"ג שוה הצלעות אשר כל אחת מצלעותיו י' אמות |
|- | |- | ||
| | | | ||
| − | : | + | ::I draw perpendicular AD on line GB. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אוציא עמוד א"ד על קו ג"ב |
|- | |- | ||
| | | | ||
| − | : | + | ::Since <math>\scriptstyle BG=BA=2\sdot BD</math>, then <math>\scriptstyle AB^2=4\sdot BD^2</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|לפי שקו ב"ג כלו' קו ב"א הוא כפל ב"ד אם כן מרובע א"ב הוא ד' כפלי מרובע ב"ד |
|- | |- | ||
| | | | ||
| − | :Therefore | + | ::Therefore, <math>\scriptstyle AD^2=3\sdot DB^2</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ולכן מרובע א"ד הוא שלשה כפלי מרובע ד"ב |
|- | |- | ||
| | | | ||
| − | : | + | ::<math>\scriptstyle GB^2=4\sdot DB^2</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ומרובע ג"ב ד' כפלי מרובע ד"ב |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Therefore, <math>\scriptstyle BG^2=\left(1+\frac{1}{3}\right)\sdot AD^2</math> |
| + | |style="text-align:right;"|א"כ מרובע ב"ג הוא חלק א' ושליש ממרובע א"ד | ||
|- | |- | ||
| | | | ||
| − | : | + | ::So, <math>\scriptstyle BG^2:AD^2=4:3</math> |
| − | + | |style="text-align:right;"|אם כן יחס מרובע ב"ג אל מרובע א"ד כיחס ד' אל ג‫' | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | : | + | ::The square of BG is multiplied by itself and the square of AD by the square of BG, so the ratio of the square of the square of BG to the square of BG by the square of AD is as the ratio of 4 to 3, which is as the ratio of 16 to 12. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ולעולם על מרובע ב"ג כלו' שיכפל [מרובע] ב"ג על עצמו ומרובע א"ד על מרבע ב"ג אם כן כח מרובע ב"ג אצל מרובע ב"ג על מרובע א"ד יחסו כיחס ד' אל ג' שהוא כיחס י"ו אל י"ב |
|- | |- | ||
| − | | | + | |colspan=2| |
| − | : | + | ::<math>\scriptstyle\left(BG^2\right)^2:\left(AD^2\sdot BG^2\right)=\left(BG^2\right)^2:\left(BG^2\sdot AD^2\right)=4:3=16:12</math> |
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | ::The square on BG by the square on AD is as the product of the rectangle on AD and BG by itself, meaning the two triangles by themselves. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{BG^2\sdot AD^2=\left(AD\sdot BG\right)^2}}</math> |
| + | |style="text-align:right;"|ומרובע ב"ג על מרובע א"ד הוא ככפל השטח הנצב הזויות אשר יקיפו בו א"ד ב"ג על עצמו כלומר שני משולשים על עצמם <s>כלומר שני משולשים על עצמם</s> | ||
|- | |- | ||
| | | | ||
| − | : | + | ::So, the ratio of the square of the square on BG to the product of the two triangles by themselves is <math>\scriptstyle16:12</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אם כן כחו של מרובע ב"ג על כפל שני משלשים על עצמם יחסם כיחס י"ו אל י"ב |
|- | |- | ||
| | | | ||
| − | : | + | ::But, the product of the two triangles by themselves is 4 times the product of one triangle by itself. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכפל שני משלשים על עצמם הוא ד' כפלי משלש א' על עצמו |
|- | |- | ||
| | | | ||
| − | : | + | ::Therefore, the ratio of the square of the square on BG to the product of one triangle by itself is <math>\scriptstyle16:3</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|‫[אם כן כחו על מרבע ב"ג אצל כפל משלש אחד על עצמו יחסו כיחס י"ו אל ג‫'] |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|אם כן גם | + | :Hence, the square of the square on BG is known, so the product of the triangle by itself is also known and therefore the triangle itself is known. |
| + | |style="text-align:right;"|אם כן כחו של מרובע ב"ג ידוע [אם כן גם תשברת המשלש על עצמו ידוע] ולכן גם המשולש עצמו ידוע | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|<big> | + | :The procedure is as follows: |
| + | |style="text-align:right;"|<big>ואופן</big> המעשה כן | ||
|- | |- | ||
| | | | ||
| − | : | + | ::Multiply 10 by itself; the result is 100. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כפול הי' על עצמם יעלו ק‫' |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Also 100 by itself; the result is 10000. |
| + | |style="text-align:right;"|עוד הק' על עצמם יעלו רבבה אחת | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::We take a third and one part of 24 from its half; it is 1875. |
| + | |style="text-align:right;"|קח מאלה שלישית מחציתם וחלק א' מכ"ד יהיה אלף תתע"ה | ||
|- | |- | ||
| | | | ||
| − | : | + | ::Extract its root, but since it does not have an expressible root, I extract its root approximately; so, its area is 43+⅓+¹/₃₈+¹/₄₀+¹/₄₁. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|קח גדרם ולפי שאין להם גדר [מדבר] אני לוקח גדרו הקרוב ויהיה התשבורת מ"ג ושליש וחלק מל"ח וחלק ממ' וחלק ממ"א |
| − | |||
|- | |- | ||
| − | | | + | |colspan=2| |
| − | + | ::<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle\sqrt{\left(\frac{1}{3}+\frac{1}{24}\right)\sdot\left[\frac{1}{2}\sdot\left(10^2\right)^2\right]}&\scriptstyle=\sqrt{\left(\frac{1}{3}+\frac{1}{24}\right)\sdot\left(\frac{1}{2}\sdot100^2\right)}\\&\scriptstyle=\sqrt{\left(\frac{1}{3}+\frac{1}{24}\right)\sdot\left(\frac{1}{2}\sdot10000\right)}=\sqrt{1875}\approx43+\frac{1}{3}+\frac{1}{38}+\frac{1}{40}+\frac{1}{41}\\\end{align}}}</math> | |
|- | |- | ||
| + | !<span style=color:Green>Right-angled triangle</span> | ||
| | | | ||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | + | *If you wish to know a right-angled triangle, which is <math>\scriptstyle\triangle ABG</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<big>אם</big> תרצה לדעת משלש נצב הזויות והוא משלש אב"ג |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*The angle at G is <math>\scriptstyle90^\circ</math> |
| + | |style="text-align:right;"|והזוית אשר אצל ג' נצבת | ||
|- | |- | ||
| | | | ||
| − | :*<math>\scriptstyle\ | + | :*The angle at A is <math>\scriptstyle\frac{2}{5}\sdot90^\circ</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואשר אצל א' שני חומשי נצבת |
|- | |- | ||
| | | | ||
| − | :: | + | :I say that the square that is generated from lines BA and AG together is five times the square on AG. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle\left(BA+AG\right)^2=5\sdot AG^2</math> |
| + | |style="text-align:right;"|ונאמר כי המרובע ההוה משני קוי ב"א א"ג ביחד הוא כפלי ה' פעמים מרובע א"ג | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::I draw AG until D. |
| + | |style="text-align:right;"|אוציא א"ג עד ד‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | ::I define <math>\scriptstyle AG=GD</math>. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואשים גם א"ג שוה לג"ד |
|- | |- | ||
| | | | ||
| − | : | + | ::I draw BD. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואגיע ב"ד |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::So, <math>\scriptstyle AB=BD</math> |
| + | |style="text-align:right;"|א"כ אם א"ב שוה לב"ד | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle\ | + | ::<math>\scriptstyle\angle ABG=\angle GBD</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואם זוית אב"ג שוה לזוית גב"ד |
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle\ | + | ::So, <math>\scriptstyle\angle GBD=\frac{3}{5}\sdot90^\circ</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אבל זוית גב"ד היא ג' חומשי נצבת |
|- | |- | ||
| | | | ||
| − | : | + | ::Since, <math>\scriptstyle\angle BAG=\frac{2}{5}\sdot90^\circ</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|בעבור שזוית בא"ג היא שני חומשי נצבת |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Hence, <math>\scriptstyle\angle ABD=\frac{6}{5}\sdot90^\circ</math> |
| + | |style="text-align:right;"|אם כן זוית אב"ד היא ו' חומשי נצבת | ||
|- | |- | ||
| | | | ||
| − | : | + | ::Therefore, <math>\scriptstyle\angle ABD</math> is an angle of a pentagon. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|א"כ זוית אב"ד <ref>54v</ref>היא זוית של בעלת ה' זויות |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle AB=BD</math> |
| + | |style="text-align:right;"|וא"ב שוה לב"ד | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Then, line AD is divided by a geometric ratio [lit. ratio of a mean and two extremes], so that the greater part is line AB. |
| + | |style="text-align:right;"|א"כ קו א"ד נחלק על יחס אמצעי ושני קצוות והחלק הגדול הוא קו א"ב | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle AG=\frac{1}{2}\sdot AD</math> |
| + | |style="text-align:right;"|וקו א"ג הוא חצי א"ד | ||
|- | |- | ||
| | | | ||
| − | : | + | :Hence, the square on lines BA and AG is five times the square on AG. |
| − | |style="text-align:right;"|אם כן | + | :<math>\scriptstyle\left(BA+AG\right)^2=5\sdot AG^2</math> |
| + | |style="text-align:right;"|אם כן המרובע ההוה משני קוי ב"א א"ג הוא [חמשה] כפלים ממרובע א"ג | ||
|- | |- | ||
| + | !<span style=color:Green>Pentagon</span> | ||
| | | | ||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | *I say: I draw pentagon ABGDH, each of its sides is 10 cubits, and I wish to find its area. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואומר אחוק מחומש אבגד"ה אשר כל אחד מצלעותיו י' אמות וארצה למצוא תשברתו |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::I determine the center of the circumscribed circle, which is Z. |
| + | |style="text-align:right;"|אקח מרכז העגולה המקפת את התמונה והיא ז‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::We draw lines ZG and ZD. |
| + | |style="text-align:right;"|ואגיע ז"ג ז"ד | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::I set perpendicular ZC on line GD. |
| + | |style="text-align:right;"|ואעמיד עמוד ז"ח על קו ג"ד | ||
|- | |- | ||
| | | | ||
| − | : | + | ::Then, <math>\scriptstyle\angle GZD=\frac{4}{5}\sdot90^\circ</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אם כן זוית גז"ד היא ד' חומשי הנצבת |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::So, <math>\scriptstyle\angle GZC=\frac{2}{5}\sdot90^\circ</math> |
| + | |style="text-align:right;"|אם כן זוית גז"ח היא ב' חומשי הנצבת | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::The angle encompassed by lines GC and CZ is a right angle. |
| + | |style="text-align:right;"|והזוית אשר יקיפוה קוי ג"ח ח"ז היא הנצבת | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Hence, <math>\scriptstyle\left(GZ+ZC\right)^2=5\sdot ZC^2</math> |
| + | |style="text-align:right;"|אם כן המרובע ההוה משני קוי ג"ז ז"ח מדובקים הם ה' כפלי מרובע ז"ח | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::But, since it is impossible to find among the numbers five times a square that is [equal to] a square, we should approximate it, so it is <math>\scriptstyle81:16</math>. |
| + | |style="text-align:right;"|אבל לפי שבמספרים לא יתכן למצוא ה' כפלי מרובע שיהיה מרובע ראוי שנקח הקרוב לו ויהיה הפ"א אל י"ו | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Then, <math>\scriptstyle\left(GZ+ZC\right):ZC=9:4</math> |
| + | |style="text-align:right;"|אם כן יחס קו ג"ז ז"ח מדובקים אל קו ז"ח כיחס ט' אל ד‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::When we separate: <math>\scriptstyle GZ:ZC=5:4</math> |
| + | |style="text-align:right;"|וכשנבדיל יהיה יחס קו ג"ז אל ז"ח כיחס ה' אל ד‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::So, <math>\scriptstyle GZ^2:ZC^2=25:16</math> |
| + | |style="text-align:right;"|אם כן יחס מרובע ג"ז אל מרובע ז"ח כיחס כ"ה אל י"ו | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::It remains that: <math>\scriptstyle GC^2:ZC^2=9:16</math> |
| + | |style="text-align:right;"|גם הנשאר מרובע ג"ח אל מרובע ז"ח כיחס ט' אל י"ו | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Hence, <math>\scriptstyle GC:ZC=3:4</math> |
| + | |style="text-align:right;"|אם כן יחס ג"ח אל ח"ז כיחס ג' אל ד‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Therefore, <math>\scriptstyle GD:ZC=6:4=3:2</math> |
| + | |style="text-align:right;"|לכן יחס ג"ד אל ז"ח כיחס ו' אל ד' שהוא כיחס ג' אל ב‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::So the ratio of the square on GD to the rectangle on GD and ZC is as the ratio of 3 to 2. |
| + | ::<math>\scriptstyle{\color{blue}{GD^2:\left(GD\times ZC\right)\approx3:2}}</math> | ||
| + | |style="text-align:right;"|א"כ יחס מרובע ג"ד אל השטח אשר יקיפו בו קוי ג"ד ז"ח כיחס ג' אל ב‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::The square on GD is known, therefore, the rectangle on GD and ZC is also known and it is twice <math>\scriptstyle\triangle GZD</math>, which is a fifth of pentagon ABGDH. |
| + | |style="text-align:right;"|ויהיה מרובע ג"ד ידוע א"כ יודע גם השטח המוקף מקוי ג"ד ז"ח והוא כפל משלש גז"ד והוא חלק חמישי מתמונת אבגד"ה בעלת חמשת זויות | ||
|- | |- | ||
| | | | ||
| − | : | + | :Hence, pentagon ABGDH is also known and its area is approximately 166⅔ cubits. |
| − | + | |style="text-align:right;"|יהיה אם כן גם תמונת אבגד"ה ידוע ויהיה תשברתו קס"ו אמות וב' שלישי אמה בקרוב | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :If another square is found that is closer to five times the other square, then we will find that the area of the shape more accurate. |
| + | |style="text-align:right;"|ואם ימצא מרובע אחר ויהיה יותר קרוב לה' כפלי מרובע אחר אז נמצא תשבורת התמונה מדוקדקת יותר | ||
|- | |- | ||
| + | !<span style=color:Green>Hexagon</span> | ||
| | | | ||
| − | |style="text-align:right;"| | + | |- |
| + | |I shall further declare the proof of the hexagon and say: | ||
| + | |style="text-align:right;"|<big>עוד</big> אודיע מופת המשושה ואומר | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :I draw hexagon ABGDHW, each of its sides is 10 cubits, and I wish to find its area. |
| + | |style="text-align:right;"|אחוק משושה עליו אבגדה"ו אשר כל אחת מצלעותיו י' אמות וארצה למצוא תשברתו | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::I determine the center of the circumscribed circle, which is C. |
| + | |style="text-align:right;"|אקח מרכז העגולה המקפת אותו והוא ח‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::We draw lines GC and CD. |
| + | |style="text-align:right;"|ונגיע ג"ח ח"ד | ||
|- | |- | ||
| | | | ||
| − | : | + | ::Then, GD is equal to both GC and CD. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|א"כ ג"ד שוה לכל אחד מן ג"ח ח"ד |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::So, <math>\scriptstyle\triangle GCD</math> is equilateral and its side is known. |
| + | |style="text-align:right;"|אם כן משולש גח"ד שוה הצלעות וצלעו ידועה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Therefore, <math>\scriptstyle\triangle GCD</math> is also known and it is one-sixth of the hexagon. |
| + | |style="text-align:right;"|אם כן גם משולש גח"ד ידוע והוא ששית חלק המשושה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|גם | + | :Hence, hexagon ABGDHW is also known. |
| + | |style="text-align:right;"|אם כן גם משושה אבגדה"ו ידוע | ||
| + | |- | ||
| + | |Since the method of the procedure is different than what we have written at first, I shall write it here: | ||
| + | |style="text-align:right;"|ובעבור שאופן המעשה בזה על פנים אחרים ממה שכתבנו תחלה אכתבנו פה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::We multiply 10 by itself; the result is 100. |
| + | |style="text-align:right;"|נכפול הי' על עצמם ההוה ק‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::100 by itself is 10000. |
| + | |style="text-align:right;"|עוד הק' על עצמם הוא רבבה | ||
|- | |- | ||
| | | | ||
| − | : | + | ::We take its quarter, which is 2500. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ונקח רביעיתם והם אלפים ב' ות"ק |
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | + | ::Multiply it by 27; the result is 67500. | |
| − | + | |style="text-align:right;"|כפלם עם כ"ז ההוה ס"ז אלפי' ות"ק | |
| − | |||
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | ::Extract its root; it is 259 and so is the area of the hexagon. | |
| − | + | |style="text-align:right;"|קח שרשם והם רנ"ט וככה תשבורת משושה | |
| − | |style=" | ||
|- | |- | ||
| + | |colspan=2| | ||
| + | ::<math>\scriptstyle{\color{blue}{\sqrt{\left[\frac{1}{4}\sdot\left(10^2\right)^2\right]\sdot27}=\sqrt{\left(\frac{1}{4}\sdot100^2\right)\sdot27}=\sqrt{\left(\frac{1}{4}\sdot10000\right)\sdot27}=\sqrt{2500\sdot27}=\sqrt{67500}\approx259}}</math> | ||
| | | | ||
| − | |||
|- | |- | ||
| − | | | + | |Proposition: if you draw a hexagon in a circle, the ratio of the line that is drawn from the center of the circle to the side of the hexagon is <math>\scriptstyle8:7</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<big>הקדמה</big> אם תחקק בעגולה תמונה בעלת ו' צלעות שוות וז' זויות הקו אשר יצא ממרכז העגולה יחסו אל צלע התמונה בעלת ז' הזויות יחס הח' אל הז‫' |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::A circle BG on the center A. |
| + | |style="text-align:right;"|ויהיה עגולת ב"ג על מרכז א‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::I draw on it a side of a hexagon, which is BG, i.e. it is equal to the line that is drawn from the center of the circle. |
| + | |style="text-align:right;"|ואחוק עליו צלע התמונה בעלת שש הזויות והוא צלע ב"ג כלו' שתהיה שוה לקו היוצא ממרכז העגולה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::I set perpendicular AD on it. |
| + | |style="text-align:right;"|ואעמיד עליה העמוד א"ד | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::So, AD is approximately equal to a side of a heptagon. |
| + | |style="text-align:right;"|אם כן יהיה א"ד בקרוב שוה <ref>55r</ref>לצלע בעלת ז' זויות | ||
|- | |- | ||
| − | |||
| | | | ||
| + | ::We draw lines BA and AG. | ||
| + | |style="text-align:right;"|ונגיע קוי ב"א א"ג | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::So, <math>\scriptstyle\triangle ABG</math> is equilateral. |
| + | |style="text-align:right;"|אם כן משלש אב"ג שוה הצלעות | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Therefore, <math>\scriptstyle AD^2=3\sdot DB^2</math> |
| + | |style="text-align:right;"|אם כן מרובע א"ד הוא ג' כפלי מרבע ד"ב | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle\left(AD:DB\right)^2\approx49:16</math> |
| + | |style="text-align:right;"|א"כ יחס ד"א אל ד"ב בכח כיחס מ"ט אל י"ו בקירוב | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle AD:DB\approx7:4</math> |
| + | |style="text-align:right;"|ובאורך יחס ד"א אל א"ב כיחס ז' אל ד‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle BG=2\sdot BD</math> |
| + | |style="text-align:right;"|ויהיה ב"ג כפל ב"ד | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Hence, <math>\scriptstyle BG:DS\approx8:7</math> |
| + | |style="text-align:right;"|אם כן יחס ב"ג אל ד"א כיחס ח' אל ז‫' | ||
|- | |- | ||
| − | !<span style=color: | + | !<span style=color:Green>Heptagon</span> |
| | | | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *If you wish to measure a heptagon: |
| + | |style="text-align:right;"|<big>ואם</big> תרצה למדוד תמונה בעלת ז' זויות | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :I draw heptagon ABGDHWZ, each of its sides is 10 cubits, and you wish to find its area. |
| + | |style="text-align:right;"|אחוק תמונת אבגדהו"ז אשר כל צלעותיו בעלי י' אמות ותרצה למצוא תשברתה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::I determine the center of the circumscribed circle, which is point T. |
| + | |style="text-align:right;"|אקח מרכז העגולה המקפת אותה והיא נקדת ט‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::I draw lines DT and TH. |
| + | |style="text-align:right;"|ואגיע קו ד"ט ט"ה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::I set perpendicular TD on line DH. |
| + | |style="text-align:right;"|ואעמיד עמוד ט"ד על קו ד"ה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::So, <math>\scriptstyle TD:DH=8:3</math> |
| + | |style="text-align:right;"|אם כן יחס ט"ד אל ד"ה כיחס ח' אל ג‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::And to DK <math>\scriptstyle8:\left(3+\frac{1}{2}\right)=16:7</math> |
| + | |style="text-align:right;"|ואל ד"כ כיחס ח' אל ג' וחצי והוא כיחס י"ו אל ז‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Therefore, <math>\scriptstyle TK:KD\approx\left(14+\frac{1}{3}\right):7=43:21</math> |
| + | |style="text-align:right;"|לכן יחס ט"כ אל כ"ד כיחס י"ד ושליש אל ז' בקרוב והוא יחס מ"ג אל כ"א | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Also, <math>\scriptstyle DH:KT\approx42:43=84:86</math> |
| + | |style="text-align:right;"|לכן גם יחס ד"ה אל כ"ט כיחס מ"ב אל מ"ג והוא יחס פ"ד אל פ"ו | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::So, the square of DH to the rectangle on DH and KT is as the same ratio. |
| + | |style="text-align:right;"|אם כן מרובע ד"ה אצל השטח הנצב הזויות אשר יקיפו בו ד"ה כ"ט הוא כזה היחס בעצמו | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Its ratio to <math>\scriptstyle\triangle DHT</math> is <math>\scriptstyle84:43</math>. |
| + | |style="text-align:right;"|אם כן יחסו אל משלש דה"ט כיחס פ"ד אל מ"ג | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Therefore, the ratio of the triangle to the heptagon is <math>\scriptstyle1:7</math> |
| + | |style="text-align:right;"|ויחס המשלש אל בעלת ז' זויות כיחס א' אל ז‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :So, the ratio of the square of DH to the heptagon is <math>\scriptstyle12:43</math> |
| + | |style="text-align:right;"|א"כ יחסו של מרובע ד"ה אל בעלת ז' זויות כיחס י"ב אל מ"ג | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Hence, the square on DH is known, therefore the heptagon is known. |
| + | |style="text-align:right;"|ומרובע ד"ה ידוע אם כן גם בעלת ז' זויות ידועה | ||
|- | |- | ||
| + | !<span style=color:Green>Octagon</span> | ||
| | | | ||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *If you wish to measure an octagon |
| + | |style="text-align:right;"|<big>ואם</big> תרצה למדוד תמונה בעלת ח' זויות | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :I draw octagon ABGDHWZC, each of its sides is 10 cubits, and you wish to find its area. |
| + | |style="text-align:right;"|אחוק תמונת אבגדהוז"ח אשר כל צלע מצלעותיה בעלת י' אמות ותרצה למצוא תשברתה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::I determine the center of the circumscribed circle, which is point K. |
| + | |style="text-align:right;"|אקח מרכז העגולה המקפת אותה והיא נקדת כ‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::I draw lines DK and HK. |
| + | |style="text-align:right;"|ואגיע קוי ד"כ ה"כ | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::I set perpendicular KL on line DH. |
| + | |style="text-align:right;"|ואעמיד עמוד כ"ל על קו ד"ה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Then, <math>\scriptstyle\angle DKH=\frac{1}{2}\sdot90^\circ</math> |
| + | |style="text-align:right;"|אם כן זויות דכ"ה היא חצי נצבת | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::So, <math>\scriptstyle\angle DKL=\frac{1}{4}\sdot90^\circ</math> |
| + | |style="text-align:right;"|לכן היא זוית דכ"ל רביע נצבת | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::I define <math>\scriptstyle\angle KDM</math> equal to it. |
| + | |style="text-align:right;"|ואעשה זוית כד"מ שוה לה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::So, <math>\scriptstyle\angle KDM=\frac{1}{4}\sdot90^\circ</math> |
| + | |style="text-align:right;"|אם כן גם זוית כד"מ רביע נצבת | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Hence, <math>\scriptstyle\angle DML=\frac{1}{2}\sdot90^\circ</math> |
| + | |style="text-align:right;"|אם כן זוית דמ"ל חצי נצבת | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::The angle at L is a right angle. |
| + | |style="text-align:right;"|והזוית אשר אצל ל' נצבת | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Then, <math>\scriptstyle DL=ML</math> |
| + | |style="text-align:right;"|אם כן קו ד"ל שוה לקו מ"ל | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::So, <math>\scriptstyle DM^2=2\sdot ML^2</math> |
| + | |style="text-align:right;"|אם כן מרובע ד"מ כפל מרובע מ"ל | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Therefore, <math>\scriptstyle DM:ML\approx17:12</math> |
| + | |style="text-align:right;"|אם כן יחס ד"מ אל מ"ל כיחס י"ז אל י"ב בקרוב | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :::<math>\scriptstyle DM=MK</math> |
| + | |style="text-align:right;"|וד"מ שוה למ"כ | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::So, <math>\scriptstyle KM:ML\approx17:12</math> |
| + | |style="text-align:right;"|אם כן יחס כ"מ אל מ"ל כיחס י"ז אל י"ב | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Therefore, <math>\scriptstyle KL:ML=KL:DL\approx29:12</math> |
| + | |style="text-align:right;"|אם כן יחס כ"ל אל מ"ל שהוא ד"ל כיחס כ"ט אל י"ב | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Hence, its ratio to DH is <math>\scriptstyle29:24</math>. |
| + | |style="text-align:right;"|אם כן יחסו אל ד"ה כיחס כ"ט אל כ"ד | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::So, the ratio of the square on DH to the rectangle on DH and KL is <math>\scriptstyle24:29</math>. |
| + | |style="text-align:right;"|אם כן יחס מרובע ד"ה אל שטח נצבת הזויות אשר יקיפו בו קוי ד"ה כ"ל כיחס כ"ד אל כ"ט | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Its ratio to <math>\scriptstyle\triangle KHD</math> is <math>\scriptstyle24:\left(14+\frac{1}{2}\right)</math>. |
| + | |style="text-align:right;"|אם כן יחסו אל משלש כה"ד כיחס כ"ד אל י"ד וחצי | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::So, its ratio to octagon ABGDHWZC is <math>\scriptstyle24:116=6:29</math> |
| + | |style="text-align:right;"|ויחסו א"כ אל תמונת אבגדהוז"ח בעלת ח' זויות כיחס כ"ד אל קי"ו שהם כיחס ו' אל כ"ט | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Hence, the square on DH is known, therefore the octagon is known. |
| + | |style="text-align:right;"|ומרובע ד"ה ידוע אם כן גם התמונה בעלת ח' זויות ידועה | ||
|- | |- | ||
| + | !<span style=color:Green>Nonagon</span> | ||
| | | | ||
| − | |||
|- | |- | ||
| − | |||
| | | | ||
| + | *If you wish to measure a nonagon: | ||
| + | |style="text-align:right;"|<big>ואם</big> רצית למדוד תמונה בעלת ט' צלעות וט' זויות | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :I draw nonagon ABGDHWZCT, each of its sides is 10 cubits, and you wish to find its area. |
| + | |style="text-align:right;"|אחוק תמונת אבגדהוזח"ט ויהיה כל צלע מצלעותיה בעל י' אמות ותרצה למצוא תשברתה | ||
|- | |- | ||
| | | | ||
| − | + | ::I draw a circumscribed circle around the shape. | |
| − | + | |style="text-align:right;"|אחוק עגולה סביב התמונה | |
| − | |||
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Its center is point L. |
| + | |style="text-align:right;"|ויהיה מרכזה נקדת ל‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::I draw HL until M. |
| + | |style="text-align:right;"|ואגיע ה"ל ואוציאנה עד מ‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::I draw MZ. |
| + | |style="text-align:right;"|ואגיע מ"ז | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::So, <math>\scriptstyle\triangle HZM</math> of the nonagon is known. |
| + | |style="text-align:right;"|אם כן משלש הז"מ מתמונת בעלת ‫<ref>55v</ref>ט' זויות ידוע | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::It has already been clarified concerning the lines of the circle that <math>\scriptstyle ZH\approx\frac{1}{3}\sdot HM</math> |
| + | |style="text-align:right;"|וכבר התבאר בקוי העגולה כי קו ז"ה הוא שלישית ה"מ בקרוב | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Therefore, <math>\scriptstyle HM^2\approx9\sdot HZ^2</math> |
| + | |style="text-align:right;"|אם כן מרובע ה"מ הוא ט' כפלי מרובע ה"ז | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::So, <math>\scriptstyle MZ^2\approx8\sdot HZ^2</math> |
| + | |style="text-align:right;"|לכן מרובע מ"ז הוא ח' כפלי מרובע ה"ז | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::But in the semicircle the angle at Z must be a right angle. |
| + | |style="text-align:right;"|ובחצי העגולה יתחייב להיות הזויות אשר אצל ז' נצבת | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Therefore, <math>\scriptstyle MZ^2:ZH^2\approx289:36</math> |
| + | |style="text-align:right;"|אם כן יחס מרובע מ"ז אל מרובע ז"ה כיחס רפ"ט אל ל"ו בקרוב | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::So, <math>\scriptstyle MZ:ZH\approx17:6</math> |
| + | |style="text-align:right;"|אם כן יחס מ"ז אל ז"ה כיחס י"ז אל ו' בקרוב | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Hence, <math>\scriptstyle ZH^2:\triangle HMZ\approx36:51=12:17</math> |
| + | |style="text-align:right;"|לכן יחס מרובע ה"ז אל משולש המ"ז כיחס ל"ו אל נ"א כלומר כיחס י"ב אל י"ז | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::So, its ratio to the nonagon is <math>\scriptstyle12:\left(76+\frac{1}{2}\right)=24:153=8:51</math> |
| + | |style="text-align:right;"|ואם כן יחסו אל התמונה בעלת ט' זויות כיחס י"ב אל ע"ו וחצי כלו' כיחס כ"ד אל קנ"ג שהם כיחס ח' אל נ"א | ||
|- | |- | ||
| − | |||
| | | | ||
| + | :Hence, the square on HZ is known, therefore the nonagon is known. | ||
| + | |style="text-align:right;"|ומרובע ה"ז ידוע אם כן גם תמונת בעלת ט' זויות ידועה | ||
|- | |- | ||
| − | + | !<span style=color:Green>Decagon</span> | |
| − | |||
| − | |||
| | | | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | *If you wish to measure a decagon: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<big>ואם</big> רצית למדוד תמונה בעלת י' זויות |
|- | |- | ||
| | | | ||
| − | : | + | :I draw decagon ABGDHWZCTI, each of its sides is 10 cubits, and you wish [to find] its area. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אחוק תמונת א'ב'ג'ד'ה'ו'ז'ח'ט'י' ויהיה כל צלע מצלעותיה בעל י' אמות ותרצה תשברתה |
|- | |- | ||
| | | | ||
| − | :: | + | ::I determine point M as the center of the circumscribed circle. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אקח מרכז העגולה המקפת אותה נקדת מ‫' |
|- | |- | ||
| | | | ||
| − | :: | + | ::I draw MH and MZ. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואגיע מ"ה מ"ז |
|- | |- | ||
| | | | ||
| − | :: | + | ::I set perpendicular MN on line HZ. |
| − | + | |style="text-align:right;"|ואעמיד עמוד מ"נ על קו ה"ז | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | : | + | ::Then, <math>\scriptstyle\angle HMZ=\frac{2}{5}\sdot90^\circ</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אם כן זוית המ"ז היא שני חומשי נצבת |
|- | |- | ||
| | | | ||
| − | :: | + | ::So, <math>\scriptstyle\angle HMN=\frac{1}{5}\sdot90^\circ</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|לכן זוית המ"נ היא חומש נצבת |
|- | |- | ||
| | | | ||
| − | :: | + | ::I define <math>\scriptstyle\angle MHS</math> equal to it. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואעמיד זוית מה"ס שוה לה |
|- | |- | ||
| | | | ||
| − | :: | + | ::So, <math>\scriptstyle\angle NSH=\frac{2}{5}\sdot90^\circ</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אם כן זוית נס"ה היא שני חומשי נצבת |
|- | |- | ||
| | | | ||
| − | :: | + | ::<math>\scriptstyle\angle HNS=90^\circ</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וזוית הנ"ס נצבת |
|- | |- | ||
| | | | ||
| − | :: | + | ::Therefore, <math>\scriptstyle HS:NS=5:4</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|[אם כן] יחס ה"ס אל נ"ס כיחס ה' אל ד‫' |
|- | |- | ||
| | | | ||
| − | :: | + | ::And its ratio to HN is as the ratio of 5 to 3. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ויחסה אל ה"נ כיחס ה' אל ג‫' |
|- | |- | ||
| | | | ||
| − | :: | + | ::HS=SM and HN=BZ |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ושוים אם ה"ס אל ס"מ ואם ה"נ אל נ"ז |
|- | |- | ||
| | | | ||
| − | :: | + | ::So, <math>\scriptstyle HZ:MN=6:9=2:3</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואם כן יחס ה"ז אל מ"נ כיחס ו' אל ט' שהם כיחס ב' אל ג‫' |
|- | |- | ||
| | | | ||
| − | :: | + | ::So, the ratio of the square on H[Z] to the rectangle on [HZ and MN] is <math>\scriptstyle2:3</math>. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וא"כ יחס מרובע ה' אל השטח נצב הזויות אשר יקיפו בו הז"מ כיחס ב' אל ג‫' |
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle | + | ::Its ratio to <math>\scriptstyle\triangle HZM</math> is <math>\scriptstyle2:3</math>. |
| − | | | + | |style="text-align:right;"|לכן יחסו אל משולש הז"מ כיחס ב' אל ג‫' |
|- | |- | ||
| − | |||
| | | | ||
| − | + | ::So, its ratio to <math>\scriptstyle\triangle HZM</math> is <math>\scriptstyle1:1+\frac{1}{2}</math>. | |
| − | + | |style="text-align:right;"|לכן יחסו אל משולש הז"מ כיחס [א'] אל א' וחצי | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | :Therefore, its ratio to the decagon is <math>\scriptstyle2:15</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|לכן יחסו אל התמונה בעלת י' זויות כיחס ב' אל ט"ו |
|- | |- | ||
| | | | ||
| − | : | + | :Hence, the square on HZ is known, therefore the decagon is known. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ומרובע ה"ז ידוע א"כ גם התמונה בעלת י' זויות ידועה |
|- | |- | ||
| + | !<span style=color:Green>Hendecagon</span> | ||
| | | | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | *If you wish to measure a hendecagon: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<big>ואם</big> רצית למדוד תמונה בעלת י"א זויות |
|- | |- | ||
| | | | ||
| − | : | + | :I draw hendecagon ABGDHWZCTIU, each of its sides is 10 cubits, and you wish to find its area. |
| − | + | |style="text-align:right;"|אחוק תמונת א'ב'ג'ד'ה'ו'ז'ח'ט'י' י"א וכל צלע מצלעותיה י' אמות ותרצה למצוא תשברתה | |
| − | |style="text-align:right;"| | ||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | ::I draw a circumscribed circle around the hendecagon, whose center is point N. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אחוק עגולה סביב התמונה אשר מרכזה יהיה נקדת נ‫' |
|- | |- | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| | | | ||
| + | ::I draw ZN until S. | ||
| + | |style="text-align:right;"|ואגיע ז"נ ואוציאנו עד ס‫' | ||
|- | |- | ||
| | | | ||
| − | + | ::I draw SC. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואגיע ס"ח |
|- | |- | ||
| | | | ||
| − | :: | + | ::So, <math>\scriptstyle\triangle ZCS</math> is ²/₁₁ of the hendecagon. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אם כן משולש זח"ס הם שני חלקים מי"א מתמונת בעלת י"א זויות |
|- | |- | ||
| | | | ||
| − | :: | + | ::It has already been clarified concerning the lines of the circle that <math>\scriptstyle ZS:ZC\approx25:7</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכבר התבאר בקוי העגולה כי יחס ז"ס ז"ח כיחס כ"ה אל ז' בקרוב |
|- | |- | ||
| | | | ||
| − | :: | + | ::Therefore: <math>\scriptstyle SC:CZ\approx24:7</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואם כן יחס ס"ח אל ח"ז כיחס כ"ד אל ז‫' |
|- | |- | ||
| | | | ||
| − | :: | + | ::So: <math>\scriptstyle ZC^2:\triangle ZCS\approx49:84=7:12</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|א"כ יחס מרובע ז"ח אל משולש זח"ס יחס מ"ט אל פ"ד שהוא כיחס ז' אל י"ב |
|- | |- | ||
| | | | ||
| − | :: | + | ::But, if the ratio of the triangle to the hendecagon is <math>\scriptstyle2:11</math>, the ratio of the square on ZC to the hendecagon is <math>\scriptstyle7:66</math> |
| − | + | |style="text-align:right;"|ואם יחס המשולש אל התמונה בעלת י"א זויות כיחס ב' אל י"א [לכן יחס מרבע ז"ח אל התמונה בעלת אחת עשרה זויות כיחס ז'] אל ס"ו | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | : | + | :Hence, the square on ZC is known, therefore the hendecagon is known. |
| − | + | |style="text-align:right;"|ומרובע ז"ח ידוע אם כן גם התמונה בעלת י"א זויות ידועה | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| + | !<span style=color:Green>Dodecagon</span> | ||
| | | | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | + | *If you wish to measure a dodecagon: | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<big>ואם</big> רצית למדוד תמונה בעלת י"ב זויות |
|- | |- | ||
| | | | ||
| − | : | + | :I draw dodecagon ABGDHWZCTIUX, each of its sides is 10 cubits, and you wish to find its area. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אחוק תמונת א'ב'ג'ד'ה'ו'ז'ח'ט'י' י"א י"ב ותהיה כל צלע מצלעותיה בעלת י' אמות ותרצה למצוא תשברתה |
|- | |- | ||
| | | | ||
| − | :: | + | ::I determine the center of the circumscribed circle, which is S. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אקח מרכז העגולה המקפת אותה והיא נקדת ס‫' |
|- | |- | ||
| | | | ||
| − | :: | + | ::I draw SC and SZ. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואגיע ס"ח ס"ז |
|- | |- | ||
| | | | ||
| − | :: | + | ::I set perpendicular SE on line HZ. |
| − | + | |style="text-align:right;"|ואעמיד עמוד ס"ע על קו ה"ז | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::Then, <math>\scriptstyle\angle ZSE=\frac{1}{6}\sdot90^\circ</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אם כן זוית זס"ע הוא ששית הנצבת |
|- | |- | ||
| | | | ||
| − | :: | + | ::I define <math>\scriptstyle\angle SZP</math> equal to it. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואעשה זוית סז"פ שוה לה |
|- | |- | ||
| − | |||
| | | | ||
| + | ::So, <math>\scriptstyle\angle ZPE=\frac{1}{3}\sdot90^\circ</math> | ||
| + | |style="text-align:right;"|אם כן זוית זפ"ע שלישית נצבת | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Then, <math>\scriptstyle PE^2=3\sdot EZ^2</math> |
| + | |style="text-align:right;"|אם כן מרובע פ"ע הוא שלישית כפלי מרובע ע"ז | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle PE:EZ\approx7:4</math> |
| + | |style="text-align:right;"|אם כן יחס פ"ע אל ע"ז כיחס ז' אל ד' בקרוב | ||
|- | |- | ||
| − | |||
| | | | ||
| + | ::<math>\scriptstyle ZC:PE\approx8:7</math> | ||
| + | |style="text-align:right;"|<ref>56r</ref>לכן גם יחס ז"ח והוא ס"פ אל פ"ע כיחס ח' אל ז' בקרוב | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle ZC:SE\approx8:15</math> |
| + | |style="text-align:right;"|לכן גם יחס ז"ח אל ס"ע כיחס [ח'] אל ט"ו | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::So the ratio of the square on ZC to the rectangle on ZC and SE is as the ratio of 8 to 15 |
| + | ::<math>\scriptstyle{\color{blue}{ZC^2:\left(ZC\times SE\right)\approx8:15}}</math> | ||
| + | |style="text-align:right;"|אם כן גם יחס מרובע ז"ח אל יחס השטח הנצב הזויות אשר יקיפו בו ז"ח ס"ע הוא כיחס [ח'] אל ט"ו | ||
|- | |- | ||
| − | |||
| | | | ||
| + | ::And its ratio to <math>\scriptstyle\triangle ZCS</math> is <math>\scriptstyle\approx8:\left(7+\frac{1}{2}\right)</math> | ||
| + | |style="text-align:right;"|ויחסו אל משולש זח"ס יהיה א"כ כיחס ח' אל ז' וחצי | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Therefore, its ratio to the dodecagon is <math>\scriptstyle\approx8:90=4:45</math> |
| + | |style="text-align:right;"|ואם כן יהיה יחסו אל התמונה בעלת י"ב זויות כיחס ח' אל צ' שהוא כיחס ד' אל מ"ה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :The square on ZC is known, therefore the dodecagon is known. |
| + | |style="text-align:right;"|ומרובע ז"ח ידוע אם כן גם תמונת בעלת י"ב זויות ידועה | ||
|- | |- | ||
| | | | ||
| − | + | ||
| + | === [The Second Section on the Division of Plane Shapes] === | ||
| + | | | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ==== The First Chapter of the Second Section of the Second Book on the Division of Plane Shapes ==== |
| + | |||
| + | |style="text-align:right;"|<big>הפרק הראשון מהחלק השני מהספר השני בחלוקת התמונו' השטחיות</big> | ||
|- | |- | ||
| − | + | |We start with the triangles. | |
| − | + | |style="text-align:right;"|<big>ונחל</big> מהמשלשים | |
|- | |- | ||
| − | | | + | |If you wish to divide an isosceles triangle into two equal parts, draw a perpendicular from one of its vertices to the base, by dividing the angle into two equal parts, so the triangle is divided into two equal triangles |
| − | |style="text-align:right;"|<big> | + | |style="text-align:right;"|<big>אם</big> רצית לחלוק משולש שוה הצלעות לשני חלקים שוים אוציא עמוד מזוית אחד מזויותיו אל התושבת וזה בשתחלוק הזוית אל שני חלקים שוים ואז יהיה המשולש נחלק לשני משלשים שוים |
|- | |- | ||
| − | | | + | |Do so if the triangle is equilateral triangle, draw a perpendicular from the vertex opposite to its base to the base. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וככה תעשה אם המשולש שוה ותוציא מזויתו שהיא מיתר התושבת עמוד אל התושבת |
|- | |- | ||
| − | | | + | |Likewise, if you want to divide it into more than two parts, divide the base into as many parts you wish and draw from each part a straight line to the vertex, meaning the top of the triangle. Then the triangle is divided as the number of parts of the base. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|גם אם רצית לחלקו ביותר משני חלקים תחלק התושבת בשיווי לחלקים שתרצה ותוציא מכל חלק מהם קו ישר אל הזוית כלומר אל ראש המשולש ואז יחלק המשולש בשעור חלקי התושבת |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :The proof of this division has already been clarified in Euclid's VI.1. |
| + | |style="text-align:right;"|ומופת זה התבאר בתמונה הא' ממאמר ו' לאקלידס | ||
|- | |- | ||
| − | |||
| | | | ||
| − | + | *Example: we wish to divide <math>\scriptstyle\triangle ABG</math> into two equal parts. | |
| − | + | |style="text-align:right;"|דמיון רצינו לחלק משולש אב"ג לשני חלקים שוים | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | :We divide the base BG into two equal parts at point D | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|חלקנו התושבת שהוא ב"ג על נקדת ד' לשני חלקים שוים |
|- | |- | ||
| | | | ||
| − | + | :We draw a perpendicular from point D to the top of the triangle, which is A, and by this it is divided into two equal triangles. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והוצאנו עמוד מנקדת ד' אל ראש המשולש שהוא א' ובזה נחלק לשני משולשים שוים |
|- | |- | ||
| | | | ||
| − | + | *If we wish to divide it into three | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואם רצינו לחלקו על ג‫' |
|- | |- | ||
| | | | ||
| − | : | + | :We divide the base into three equal parts, which ar AD, DH, HG. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|חלקנו התושבת על ג' חלקים שוים והם א"ד ד"ה ה"ג |
|- | |- | ||
| | | | ||
| − | : | + | :Then, we draw DA and HA, and by this it is divided into three equal parts. |
| − | + | |style="text-align:right;"|אחר הוצאנו ד"א ה"א ובזה נחלק על שלשה חלקים שוים | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| − | | | + | |Do the same if you wish to divide it into more than that. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|גם ככה תעשה אם רצית לחלקו יותר מזה |
|- | |- | ||
| − | | | + | |If you wish to divide the triangle mentioned into two equal parts, so that one part is a triangle and the other part is a trapezium. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אמנם אם רצית לחלק המשולש הנזכר לב' חלקים שוים מהצד האחד עד שיהיה החלק משולש והאחד נוטה |
|- | |- | ||
| − | |||
| | | | ||
| + | *As <math>\scriptstyle\triangle ABG</math>: we divide it into <math>\scriptstyle\triangle ADH</math> and trapezium DHBG. | ||
| + | |style="text-align:right;"|כגון משולש אב"ג ונחלקהו על משולש אד"ה ועל נוטה ד"הב"ג | ||
|- | |- | ||
| | | | ||
| − | + | :We do as follows: | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|נעשה כך |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :We divide one side at a point such that the square of one part is twice the square of the other part. |
| + | |style="text-align:right;"|נחלק הצלע האחד על נקדה שיהיה מרובע החלק האחד כפל מרובע החלק השני | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :We draw a line from this point parallel to the base of the triangle and join it with the other side. |
| + | |style="text-align:right;"|ונוציא מהנקודה ההיא קו נכוחי לתושבת המשולש ונגיעהו על הצלע האחר | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Then the triangle is divided into two equal parts: <math>\scriptstyle\triangle ADH</math> and trapezium DHBG. |
| + | |style="text-align:right;"|ואז נחלק המשלש לשני חלקים שוים משלש אד"ה ונוטה ד"הב"ג | ||
| + | |- | ||
| + | |Euclid has already explained the proof of this in Book 6, proposition 18: that for every two similar triangles, the ratio of the triangle to the triangle is as the duplicate ratio of its side to its corresponding side. | ||
| + | |style="text-align:right;"|והמופת על זה כבר ביאר אקלידס ב[[ספר_היסודות_לאקלידס#Elements_VI_18|תמונת י"ח ממאמר ו']] שכל שני משלשים דומים יחס המשולש אל המשולש כיחס צלעו אל צלעו הנכחי לו שנוי | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :It is known that the larger triangle, which is <math>\scriptstyle\triangle ABG</math>, is similar the the smaller triangle, which is <math>\scriptstyle\triangle ADH</math>. |
| + | |style="text-align:right;"|וידוע שהמשלש הגדול והוא המשולש אב"ג דומה אל המשולש הקטן והוא משולש אד"ה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*For, the angle at [A] is shared by the two triangles. |
| + | |style="text-align:right;"|כי הזוית אשר אצל ח' משותפת לשני המשולשי‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*<math>\scriptstyle\measuredangle ADH=\measuredangle ABG</math>, as they are two corresponding angles between parallel lines, when a straight line falls on them, and the exterior angle is equal to its corresponding angle. |
| + | |style="text-align:right;"|וזוית אד"ה שוה לזוית אב"ג כי הם שתי זויות המומרות אשר על קוים נכוחיים כשנפל עליהם קו ישר והחיצונה ‫<ref>56v</ref>שוה לשכנגדה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*Similarly, <math>\scriptstyle\measuredangle AHD=\measuredangle AGB</math>, for the same reason itself. |
| + | |style="text-align:right;"|גם ככה זוית אה"ד שוה לזוית אג"ב לזה הענין עצמו | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :So, the sides on the equal angles are reciprocally proportional: |
| + | |style="text-align:right;"|אם כן מיתרי הזויות השוות מתיחסות | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :<math>\scriptstyle AB:AD=AH:HG=DH:BG</math> |
| + | |style="text-align:right;"|יחס א"ב אל א"ד כיחס א"ה אל ה"ג וכיחס ד"ה אל ב"ג | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Therefore, the two triangles are similar. |
| + | |style="text-align:right;"|אם כן שני המשולשים דומים | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Thus: <math>\scriptstyle\triangle ABG:\triangle ADH=\left(AB:AD\right)^2</math> |
| + | |style="text-align:right;"|ולכן יהיה יחס משלש אב"ג אל משלש אד"ה כיחס צלע א"ב אל צלע א"ד שנוי | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :From [Elements, VI.19], this ratio itself is: <math>\scriptstyle AB^2:AD^2</math>. |
| + | |style="text-align:right;"|וזה היחס מי"ו מח' בעצמו הוא יחס צלע מרובע א"ב אל מרובע צלע א"ד | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Because, when you multiply the ratio of the side to the side by itself, and this is what is called "the duplicate ratio", the result is the ratio of the square [of the side] to the square [of the side]. |
| + | |style="text-align:right;"|כי כאשר תכפול יחס הצלע אל הצלע בעצמו והוא אשר יקרא היחס שנוי יהיה הנולד יחס המרבע אל מרבע | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*Example: if AB is 6 cubits and AD is 2 cubits. |
| + | |style="text-align:right;"|דמיון אם צלע א"ב ו' אמות וצלע א"ד ב' אמות | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::The ratio of 2 to 6 is as the ratio of the third to one. |
| + | ::<math>\scriptstyle{\color{blue}{AD:AB=2:6=\frac{1}{3}:1}}</math> | ||
| + | |style="text-align:right;"|יהיה יחס ב' אל ו' כיחס השלישית אל האחד | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::When you take the duplicate ratio of the third to one, it is as you multiply the third by the the third; the result is a ninth and it is the ratio of the small triangle to the larger triangle. |
| − | + | ::<math>\scriptstyle{\color{blue}{\left(AD:AB\right)^2=\left(\frac{1}{3}:1\right)^2=\frac{1}{3}\sdot\frac{1}{3}=\frac{1}{9}}}</math> | |
| − | + | |style="text-align:right;"|וכאשר תקח יחס השלישית אל האחד שנוי וזה כשתכפול השלישית על השלישית יהיה הנולד תשיעית וזהו יחס המשלש הקטן אל המשלש הגדול | |
|- | |- | ||
| | | | ||
| − | + | ::Also, when you take the square of 6, which is one side; it is 36. The square of 2, which is the side of the other triangle, is 4. The ratio of 4 to 36 is the ratio of the ninth to one and it is [the ratio of] the small triangle to the larger triangle. | |
| − | + | ::<math>\scriptstyle{\color{blue}{AD^2:AB^2=2^2:6^2=4:36=\frac{1}{9}:1}}</math> | |
| − | + | |style="text-align:right;"|גם ככה כשתקח מרובע ו' שהוא הצלע האחד הוא ל"ו ומרובע ב' שהוא צלע המשולש האחר ד' יהיה יחס ד' אל ל"ו יחס התשיעית אל האחד והוא המשלש הקטן אל הגדול | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Therefore, when you want to divide the triangle into two equal parts, we divide AB at point D, so that <math>\scriptstyle AB^2=2\sdot AD^2</math> |
| + | |style="text-align:right;"|על כן כשתרצה לחלק המשולש לשני חלקים שוים נחלק צלע א"ב על נקודה והיא ד' עד שיהיה מרובע צלע א"ב כפל מרובע א"ד | ||
|- | |- | ||
| − | |||
| | | | ||
| + | :Once we have done this, we draw a line parallel to BG from D, which is DH. | ||
| + | |style="text-align:right;"|וכאשר עשינו כן הוצאנו מנקדת ד' קו נכחי לקו ב"ג והוא ד"ה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :By that the triangle is divided into two equal parts: one part is <math>\scriptstyle\triangle ADH</math> and the other is trapezium DHBG. |
| + | |style="text-align:right;"|ובזה נחלק המשלש לשני חלקים שוים החלק האחד משלש אד"ה והשני נוטה ד"הב"ג | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*Example: take from point A, which is the top of the triangle, 5 parts of 7 of it, minus a tenth of a seventh. |
| + | |style="text-align:right;"|דמיון שתקח מנקדת א' אשר היא ראש המשולש ה' חלקים מז' בו פחות עשירית השביעית | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::For instance: line AB is 7 cubits and its square is 49. |
| + | ::<math>\scriptstyle{\color{blue}{7^2=49}}</math> | ||
| + | |style="text-align:right;"|המשל שקו א"ב ז' אמות ומרובעו מ"ט | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::We measure 5 cubits minus a tenth of a seventh from point A and mark a point there. |
| + | |style="text-align:right;"|ונמדוד מנקדת א' ה' אמות פחות עשירית השביעית ונסמן שם נקדה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::The square of the line from point A to the point we marked is 24 cubits and a half and it is half the area of the great triangle. |
| + | ::<math>\scriptstyle{\color{blue}{\left[5-\left(\frac{1}{10}\sdot\frac{1}{7}\right)\right]^2\approx24+\frac{1}{2}}}</math> | ||
| + | |style="text-align:right;"|ויהיה מרובע הקו אשר הוא מנקדת א' עד הנקדה אשר סמננו כ"ד אמות וחצי והוא מחצית התשבורת של המשלש הגדול | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|<big> | + | ::We know from this that the smaller triangle is half the great triangle and the remaining trapezium is its other half. |
| + | |style="text-align:right;"|ידענו מזה שהמשולש הקטן הוא חצי המשולש הגדול ונשאר הנוטה חציו אחר | ||
| + | |- | ||
| + | |If you wish to divide it into three [equal] parts, so that they are two trapezoids and one triangle: | ||
| + | |style="text-align:right;"|<big>ואם</big> רצית לחלקו לג' חלקים עד שיהיו שני נוטים ומשולש א‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Divide the long side into six, then count about 5 and a fifth from point A, which is the top of the triangle and mark a point there, which is B. |
| + | |style="text-align:right;"|חלק צלע הגדול על ו' ומנה מנקדת א' שהוא ראש המשולש ה' וחמישית בקרוב וסמן שם נקדה והיא ב‫' | ||
|- | |- | ||
| − | |||
| | | | ||
| + | :Draw a line from this point parallel to the base of the great triangle; it is BG. | ||
| + | |style="text-align:right;"|והוצא מזאת הנקודה קו נכחי לתושבת המשולש הגדול והוא ב"ג | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :It is divided then into a smaller triangle and a trapezoid. |
| + | |style="text-align:right;"|והנה יחלק אל משלש קטן ואל נוטה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :The smaller [triangle] is a third of the larger triangle. |
| + | |style="text-align:right;"|ויהיה הקטן שלישית המשולש הגדול | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Divide also the trapezoid into halves, by that we divide the base of the great triangle into two equal parts and mark a point there, which is Z. |
| + | |style="text-align:right;"|עוד תחלק הנוטה בשני חצאים וזה כשנחלק תושבת המשולש הגדול לשני חלקים שוים ונסמן שם נקדה והיא ז‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :We also divide the base of the smaller triangle this way and draw a straight line from point to point. |
| + | |style="text-align:right;"|עוד נחלק תושבת המשולש הקטן כן ונגיע קו ישר מנקדה אל נקדה | ||
|- | |- | ||
| − | |||
| | | | ||
| + | :Then the trapezoid is divided into two equal parts. | ||
| + | |style="text-align:right;"|אז נחלק הנוטה לשני חלקים שוים | ||
| + | |- | ||
| + | |If you wish to divide it into two equal triangles and one trapezoid: | ||
| + | |style="text-align:right;"|<big>ואם</big> רצית לחלקו לשני משלשים שוים ולנוטה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|< | + | :Count a little less than 6 parts of 9 of the side from the top of the triangle, which is point A, and mark point D there. |
| + | |style="text-align:right;"|מנה מראש המשולש אשר הוא [א' ו']‫<ref>P1031 om.</ref> חלקים פחות מעט מט' בצלע וסמן שם נקדת ד‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Draw a line from point D parallel to the base of the great triangle; it is DH. |
| + | |style="text-align:right;"|והוצא נקדת קו נכחי מנקדת ד' אל תושבת המשולש הגדול והוא קו ד"ה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :The smaller triangle is two parts of the larger triangle. |
| + | |style="text-align:right;"|ויהיה המשלש הקטן שני חלקים מהמשולש הגדול | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :The third part is the remaining trapezoid DHBG. |
| + | |style="text-align:right;"|ונשאר נוטה ד"הב"ג החלק השלישי | ||
|- | |- | ||
| − | |||
| | | | ||
| + | :<math>\scriptstyle\triangle ADH</math> is two parts: | ||
| + | |style="text-align:right;"|ומשלש אד"ה שני חלקים | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Divide the base DH into halves at point Z and draw a line from it to the top of the triangle. |
| + | |style="text-align:right;"|תחלק תושבת ד"ה לחצאים על נקדת ז' והוצא ממנו קו אל ראש המשולש | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :So the small triangle is divided into two and we find that the whole large triangle is divided into three equal parts: the two triangles <math>\scriptstyle\triangle ADZ</math> <math>\scriptstyle\triangle AZH</math> and the trapezoid DHBG. |
| + | |style="text-align:right;"|הנה נחלק המשלש הקטן לשנים ונמצא כל המשולש הגדול נחלק לג' ‫<ref>57r</ref>חלקים שוים לשני משלשי אד"ז אז"ה ולנוטה ד"הב"[ג]‫<ref>P1031 ה</ref> | ||
| + | |- | ||
| + | |This is regarding to an equilateral triangle, or an isosceles triangle, whose different side is the base of the triangle. | ||
| + | |style="text-align:right;"|וזה במשלש שוה הצלעות או שוה השוקים שתהיה צלע המתחלפ' תושבת המשולש | ||
| + | |- | ||
| + | |If it is a scalene triangle, or an isosceles triangle, of which the different side is not the base of the triangle and you wish to divide it into two equal parts: | ||
| + | |style="text-align:right;"|<big>ואם</big> היה המשולש [מתחלף]‫<ref>P1031 שוה</ref> הצלעות או שוה השוקים ולא תהיה הצלע המתחלפת תושבת המשולש ותרצה לחלקו בשני חלקים שוים | ||
|- | |- | ||
| − | |||
| | | | ||
| + | *Example: <math>\scriptstyle\triangle ABG</math> a scalene triangle, or <math>\scriptstyle\triangle DHZ</math> an isosceles triangle, but the base is not the different side, and we want to divide them into two or three parts: | ||
| + | |style="text-align:right;"|דמיון משלש אב"ג מתחלף הצלעות או משולש ד[ה]‫<ref>P1031 א</ref>"ז שוה השוקים ואין התושבת צלע המתחלפת ונרצה לחלקם בשני חלקים או בג‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Find the area of the whole triangle, as I taught you in the measuring of the triangles. |
| + | |style="text-align:right;"|תמצא תשבורת כל המשולש כמו שהודעתיך במדידת המשולשים | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Then, mark a point on one of its sides and we divide it into a right-angled triangle and a trapezoid, so that the area of the right-angled triangle is its half or its third, according to what you wish and the rest is the trapezoid. |
| + | |style="text-align:right;"|אח"כ תסמן בצלעו האחת נקדה ונחלקהו אל משולש נצב הזויות ואל נוטה עד שיהיה תשבורת המשולש נצב הזויות חציו או שלישיתו כפי מה שתבקש והשאר ישארו הנוטה | ||
|- | |- | ||
| − | | | + | |I have already taught you the measurement of all these. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכבר הודעתיך מדידת כל אלה |
|- | |- | ||
| − | |||
| | | | ||
| + | |||
| + | ==== The Second Chapter on the Division of the quadrilateral and the Circle ==== | ||
| + | |||
| + | |style="width:45%; text-align:right;"|<big>הפרק השני</big> בחלוקת התמונות המרובעות והעגולה | ||
|- | |- | ||
| − | | | + | |You already know the types of the quadrilateral shapes and how they are divided into square, rhombus, parallelogram and scalene quadrilateral; and the scalene quadrilaterals are divided into various types. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כבר ידעת מיני התמונות המרובעות ואיך יחלק אל שוה הצלעות נצב הזויות ואל שוה הצלעות ובלתי נצב הזויות ואל מרובע ארוך בלתי נצב הזויות שוה הצלעות הנכחיות ואל נוטה והנוטה מינים רבים |
|- | |- | ||
| + | !<span style=color:Green>Square</span> | ||
| | | | ||
| − | |||
|- | |- | ||
| − | + | |I shall start with the square, as it precedes them by nature and by degree | |
| − | + | |style="text-align:right;"|<big>ואחל</big> מהמרובע שוה הצלעות נצב הזויות כי הוא הקודם בטבע ומעלה מהם | |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *I say that if you wish to divide the square ABGD into two [equal] parts: |
| + | |style="text-align:right;"|ואומר אם תרצה לחלק מרבע אבג"ד שוה הצלעות נצב הזויות לשני חלקי‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Divide DG into two halves at point Z, and draw a line from point Z parallel to each of the sides AD and BG, hence the square is divided into two equal part |
| + | |style="text-align:right;"|תחלק צלע ד"ג לשני חצאים על נקדת ז' ותוציא מנקדת ז' צלע נכחי לכל אחת מצלעות א"ד ב"ג ובזה יחלק המרובע לשני חלקים שוים | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|<big> | + | :The proof of this division: |
| + | |style="text-align:right;"|<big>והמופת</big> על זה החלוק | ||
|- | |- | ||
| | | | ||
| − | : | + | :It has already been clarified in Euclid's VI.1 that the ratio of the sides of the parallelograms, whose heights are the same magnitude, to one another is as the ratio of their bases to one another. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כבר התבאר ב[[ספר_היסודות_לאקלידס#Elements_VI_1|תמונת א' ממאמר ו' מאקלידס]] שהצלעות נכחיי הצלעות כאשר היה גבהותם בשעור אחד הנה יחס קצתם אל קצתם כיחס תושבותיהם קצתם אל קצת |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :<math>\scriptstyle DG:ZG=2\sdot AB</math> |
| + | |style="text-align:right;"|ויחס ד"ג אל ז"ג כפל א"ב | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle | + | :*<math>\scriptstyle DZ=\frac{1}{2}\sdot DG</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ד"ז מחצית ד"ג |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*<math>\scriptstyle ZG=\frac{1}{2}\sdot DG</math> |
| + | |style="text-align:right;"|גם ככה ז"ג מחצית ד"ג | ||
|- | |- | ||
| − | |||
| | | | ||
| + | ::According to this proof itself it can also be divided from AD to BG. | ||
| + | |style="text-align:right;"|ובזה המופת בעצמו יחלק גם מצלע א"ד אל ב"ג | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Do the same if you wish to divide it into three parts or more. |
| + | |style="text-align:right;"|וכן תעשה אם רצית לחלקו לג' חלקים ויותר | ||
|- | |- | ||
| | | | ||
| − | + | *If you want to divide it from the vertices | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואם רצית לחלקו מהזויות |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Connect vertex A to vertex G, so the square is divided into two [equal] parts. |
| + | |style="text-align:right;"|תגיע זוית א' אל זוית ג' ויחלק המרבע לשנים חלקים | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle | + | :The proof of this division: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<big>והמופת</big> על החלוקה הזאת |
| − | | | + | |- |
| − | + | | | |
| + | :<math>\scriptstyle\triangle ABG=\triangle AGD</math> | ||
| + | |style="text-align:right;"|כי שני משלשי אב"ג אג"ד שוים | ||
| + | |- | ||
| + | | | ||
| + | :*<math>\scriptstyle AB=DG</math> | ||
| + | |style="text-align:right;"|כי צלע א"ב שוה לצלע ד"ג | ||
|- | |- | ||
| | | | ||
| − | + | :*<math>\scriptstyle BG=AD</math> | |
| − | + | |style="text-align:right;"|וצלע ב"ג לצלע א"ד | |
| − | |||
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*<math>\scriptstyle\measuredangle ABG=\measuredangle ADG</math> |
| + | |style="text-align:right;"|וזוית אב"ג שוה לזוית אד"ג | ||
|- | |- | ||
| − | |||
| − | |||
| | | | ||
| + | :*The base is shared by both. | ||
| + | |style="text-align:right;"|והתושבת משותפת | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :So the two triangles are equal. |
| + | |style="text-align:right;"|אם כן שני המשולשים שוים | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *If you wish to divide it into three equal parts: |
| + | |style="text-align:right;"|<big>ואם</big> רצית לחלקו לשלשה חלקים שוים | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :By the first way - you already know the way. |
| + | |style="text-align:right;"|בדרך הראשונה כבר ידעת דרכו | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :By the second way, do as follows: |
| + | |style="text-align:right;"|אמנם בזאת הדרך השנית תעשה כך | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Take 2-thirds of the side and join the point with the vertex. |
| + | |style="text-align:right;"|תקח מהצלע ב' שלישיו ותגיע הזוית עד הנקודה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Do the same with the other side, then the square is divided into three equal parts. |
| + | |style="text-align:right;"|וככה תעשה מהצלע האחר ויחלק המרובע לג' חלקי' שוים | ||
|- | |- | ||
| − | |||
| − | |||
| | | | ||
| + | *Example: we wish to divide square ABGD into three [equal] parts through the vertices. | ||
| + | |style="text-align:right;"|דמיון רצינו לחלק מרבע אבג"ד לשלשה חלקים מדרך הזויות | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Let each of its side by 10 cubits. |
| + | |style="text-align:right;"|ויהיה כל צלע מצלעותיו י' אמות | ||
|- | |- | ||
| − | |||
| − | |||
| | | | ||
| + | :We take from DG 6 parts and two-thirds of one part at point H, then we draw AH, so the right-angled <math>\scriptstyle\triangle ADH</math> is a third of the square. | ||
| + | |style="text-align:right;"|לקחנו מצלע ד"ג ו' חלקים ושני שלישי חלק עד נקדת ה' והגענו א"ה והנה משולש ‫<ref>57v</ref>אד"ה נצב הזויות לשלישית המרובע | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :We do the same from point B: we divide it into 6 parts and two-thirds of one part at point Z, then we draw GZ, so the right-angled <math>\scriptstyle\triangle GZB</math> is one-third [of the square]. |
| + | |style="text-align:right;"|גם ככה עשינו מנקדת ב' חלקנוהו ו' חלקים ושני שלישי חלק עד נקדת ז' והגענו ג"ז והנה משולש גז"ב נצב הזויות השלישית האחד | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :The remaining parallelogram AHZG is the other third. |
| + | |style="text-align:right;"|ונשאר אהז"ג הנכחי הצלעות השלישית האחר | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :The proof of this division: |
| + | |style="text-align:right;"|<big>והמופת</big> על זה החלוק | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :It has already been clarified in Euclid's I.[41] regarding the parallelograms and the triangles that are on the same bases and are between the same two parallel lines, that the parallelogram is double the triangle. |
| + | |style="text-align:right;"|כבר התבאר ב[[ספר_היסודות_לאקלידס#Elements_I_41|תמונת ל"ו ממאמר א' מאיקלידס]] שהשטחים הנכחיי הצלעות מהמשולשים שהם על תושבות שוות ובמה שבין שני קוים נכוחיים יהיה השטח הנכחי הצלעות כפל המשולש | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :The base of AZHG is half the base of <math>\scriptstyle\triangle GBZ</math>, but they should be equal. |
| + | |style="text-align:right;"|והנה תושבת אזה"ג מחצית תושבת משולש גב"ז וראוי שיהיו שוים | ||
|- | |- | ||
| − | |||
| − | |||
| | | | ||
| + | :AZHG is equal to the rectangle BTHG, because they have the same base and are between the same two parallel lines. | ||
| + | |style="text-align:right;"|ועוד כי שטח אזה"ג שוה לשטח בטה"ג הנכחי הצלעות ונצב הזויות כי הם תושבת אחת ובמה שבין קוים הנכוחיים | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :[BTHG] is a third of the square ABGD, because this is the ratio of its base to its base. |
| + | |style="text-align:right;"|ושטח אזה"ג שלישית מרובע אבג"ד כי כן יחס תושבתו אל תושבתו | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Therefore AZHG is a third of the whole square. |
| + | :<math>\scriptstyle AZHG=BTHG=\frac{1}{3}\square ABGD</math> | ||
| + | |style="text-align:right;"|א"כ שטח אזה"ג שלישית כל המרובע | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :The two triangles are equal: |
| + | |style="text-align:right;"|ושני המשולשים שוים | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*<math>\scriptstyle AD=GB</math> |
| + | |style="text-align:right;"|כי צלע א"ד כמו צלע ב"ג | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*<math>\scriptstyle DH=BZ</math> |
| + | |style="text-align:right;"|וצלע ד"ה כמו צלע ב"ז | ||
|- | |- | ||
| − | |||
| − | |||
| | | | ||
| + | :*<math>\scriptstyle\measuredangle ADH=\measuredangle GBZ</math> | ||
| + | |style="text-align:right;"|וזוית אד"ה שוה לזוית גב"ז | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :So, square ABGD is divided into three equal parts. |
| + | |style="text-align:right;"|הנה אם כן מרובע אבג"ד נחלק לג' חלקים שוים | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *If you wish to divide it into four [equal] parts: |
| + | |style="text-align:right;"|<big>ואם</big> רצית לחלקו לד' חלקים | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :According to the first way - you can divide it by two ways: |
| + | |style="text-align:right;"|אם מן הדרך הראשונה תוכל לחלקו בשני אופנים | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :The first is that you divide the side of the square into four equal parts: AH, HZ, ZC, CB, then you draw HT, ZK, CL, so the square is divided into four equal parts. |
| + | |style="text-align:right;"|האחד שתחלק צלע המרובע על ד' חלקים שוים על א"ה ועל ה"ז ועל ז"ח ועל ח"ב ותגיע ה"ט ז"כ ח"ל ויחלק המרובע לד' חלקים שוים | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :The proof of this division: |
| + | |style="text-align:right;"|<big>והמופת</big> על החלוקה הזאת | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :I have explained it in its division into two parts. |
| + | |style="text-align:right;"|בארתיו בחלוקתו לשני חלקים | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|תם ונשלם<ref>NY 2639: נשלם הספר חכמת המספר וחכמת המדות שחבר מרנו ורבנו החכם ב"ר מרדכי כומטיינו יצ"ו בהכ"ר אליעזר כומטיינו יעמ"ש ונשלם ע"י לי שבתי בכ"ר משה ז"ל מקנדייה שנת הרל"ח בכ"ד לחדש אדר שבח לאל יתעלה אשר לו התהלה והיכולת וזאת היא ההעתקה השנית וכתבתיהו לר' כלב המכונה אפדופילו י"ל בכ"ר אליהו יצ"ו בכ"ר יהודה</ref> | + | :The second way is that you divide it lengthwise into two [equal] parts, then divide it also breadthwise, so it is divided into four equal parts. |
| + | |style="text-align:right;"|<big>והאופן</big> השני שתחלקהו מהאורך לשני חלקים עוד מהרחב תחלקהו ויחלק לד' חלקים שוים | ||
| + | |- | ||
| + | | | ||
| + | *Example: we wish to divide square ABGD into four [equal] parts. | ||
| + | |style="text-align:right;"|דמיון רצינו לחלק מרבע אבג"ד לד' חלקים | ||
| + | |- | ||
| + | | | ||
| + | :We divide AB into two [equal] parts at point H and draw HZ, so it is parallel to AD and BG. The square is divided into two [equal] parts. | ||
| + | |style="text-align:right;"|חלקנו צלע א"ב לשני חלקים על נקדת ה' והגענו ה"ז ויפול נכחי לא"ד ולב"ג ויחלק המרובע לב' חלקים | ||
| + | |- | ||
| + | | | ||
| + | :We also divide AD into two at point C and draw CT, so it is parallel to AB and DG. The square is divided into four [equal] parts. | ||
| + | |style="text-align:right;"|עוד חלקנו צלע א"ד לשנים על נקדת ח' והגענו [ח"ט] ויפול נכחי לא"ב ולד"ג ויחלק המרובע על ד' חלקים | ||
| + | |- | ||
| + | | | ||
| + | :The proof of this division: | ||
| + | |style="text-align:right;"|<big>והמופת</big> על החלוקה הזאת | ||
| + | |- | ||
| + | | | ||
| + | :<math>\scriptstyle\square AHCB=\square CBDZ</math> | ||
| + | |style="text-align:right;"|כי מרובע אהח"ב שוה למרובע חבד"ז | ||
| + | |- | ||
| + | | | ||
| + | :*<math>\scriptstyle AH=AC</math> | ||
| + | |style="text-align:right;"|כי צלע א"ה שוה לצלע א"ח | ||
| + | |- | ||
| + | | | ||
| + | ::Because when you subtract equals from equals the remainders are equal. | ||
| + | |style="text-align:right;"|כי כשתחסר מן השוה שוה יהיה הנשאר שוה | ||
| + | |- | ||
| + | | | ||
| + | ::And [it is equal] to CK and KH, because they are parallel. | ||
| + | |style="text-align:right;"|ולצלע ח"כ וכ"ה כי הם נכחיי הצלעות | ||
| + | |- | ||
| + | | | ||
| + | :*The same for the remaining three squares. | ||
| + | |style="text-align:right;"|וכן לג' המרובעים הנשארים | ||
| + | |- | ||
| + | | | ||
| + | :Therefore, square ABGD is divided into four [equal] squares that are similar to square ABGD. | ||
| + | |style="text-align:right;"|אם כן כל מרובע אבג"ד נחלק לד' מרובעים דומים למרובע אבג"ד | ||
| + | |- | ||
| + | | | ||
| + | *If you wish to divide it into [four equal] parts by the other way, i.e. through the vertices: | ||
| + | |style="text-align:right;"|<big>ואם</big> רצית לחלקו לג' חלקים מדרך השנית כלומר מדרך הזויות | ||
| + | |- | ||
| + | | | ||
| + | :Draw its two diagonals, so it is divided into four equal parts, each part is an isosceles triangle. | ||
| + | |style="text-align:right;"|תגיע ב' אלכסוניו ויחלק לד' חלקים שוים וכל חלק יהיה משולש שוה השוקים | ||
| + | |- | ||
| + | | | ||
| + | *Example: we wish to divide square ABGD into four equal parts through the vertices. | ||
| + | |style="text-align:right;"|דמיון רצינו לחלק מרבע אבג"ד לד' חלקים שוים מדרך הזויות | ||
| + | |- | ||
| + | | | ||
| + | :We draw diagonal AG and diagonal BD, so the square is divided into four triangles: <math>\scriptstyle\triangle AHB</math>, <math>\scriptstyle\triangle BHG</math>, <math>\scriptstyle\triangle GHD</math>, <math>\scriptstyle\triangle DHA</math>. | ||
| + | |style="text-align:right;"|הוצאנו אלכסון א"ג ואלכסון ב"ד ויחלק המרובע לד' משולשים משולש אה"ב ומשולש בה"ג ומשולש גה"ד ומשולש דה"א | ||
| + | |- | ||
| + | | | ||
| + | :<math>\scriptstyle\triangle AHB=\triangle DHG</math> | ||
| + | |style="text-align:right;"|והנה משולש אה"ב שוה למשולש דה"ג | ||
| + | |- | ||
| + | | | ||
| + | :*<math>\scriptstyle\measuredangle AHB=\measuredangle DHG</math> | ||
| + | |style="text-align:right;"|כי זוית אה"ב לזוית דה"ג | ||
| + | |- | ||
| + | | | ||
| + | :*<math>\scriptstyle\measuredangle ABH=\measuredangle HDG</math> | ||
| + | |style="text-align:right;"|וזוית אב"ה שוה לזוית הד"ג | ||
| + | |- | ||
| + | | | ||
| + | :*<math>\scriptstyle AB=DG</math> | ||
| + | |style="text-align:right;"|וצלע א"ב שוה לצלע ד"ג בעצמו | ||
| + | |- | ||
| + | | | ||
| + | :<math>\scriptstyle\triangle AHD=\triangle BHG</math> | ||
| + | |style="text-align:right;"|ולזה ישוה משולש אה"ד אל משולש בה"ג | ||
| + | |- | ||
| + | | | ||
| + | :<math>\scriptstyle\triangle AHD=\triangle AHB</math> | ||
| + | |style="text-align:right;"|ומשולש אה"ד ישוה למשולש אה"ב | ||
| + | |- | ||
| + | | | ||
| + | :*<math>\scriptstyle AD=AB</math> | ||
| + | |style="text-align:right;"|כי צלע א"ד ישוה לצלע א"ב | ||
| + | |- | ||
| + | | | ||
| + | :*AB is shared by both. | ||
| + | |style="text-align:right;"|וצלע א"ב משותף | ||
| + | |- | ||
| + | | | ||
| + | :*<math>\scriptstyle\measuredangle AHB=\measuredangle AHD</math> as both are right angles. | ||
| + | |style="text-align:right;"|וזוית אה"ב שוה לזוית אה"ד כי שתיהן נצבות | ||
| + | |- | ||
| + | | | ||
| + | :My saying that they are isosceles is because the side of the square is greater than half the diagonal and all the halves of the diagonals are equal. | ||
| + | |style="text-align:right;"|ואמרי שהם שוה השוקים כי צלע המרובע הגדול מחצית הקטר וחציי הקטרים הכל שוים | ||
| + | |- | ||
| + | |We have already explained the rules of division of the square [lit. the equilateral right angled quadrangle]. | ||
| + | |style="text-align:right;"|‫<ref>58r</ref>הנה כבר בארנו משפטי חלוקת המרובע השוה הצלעות נצב הזויות | ||
| + | |- | ||
| + | !<span style=color:Green>Rectangle</span> | ||
| + | | | ||
| + | |- | ||
| + | |The same rule itself is applied to the rectangle, like this, because it is similar to it in every way and its proofs are as its proofs. | ||
| + | |style="text-align:right;"|וזה המשפט בעצמו אל הנכחי הצלעות הנצב הזויות הנקרא ארוך כזה כי הוא שוה עמו בכל הדרכים ומופתיו כמופתיו | ||
| + | |- | ||
| + | !<span style=color:Green>Rhombus</span> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | *If you wish to divide an equilateral quadrilateral that is not right-angled, like this, into two [equal] parts: | ||
| + | |style="text-align:right;"|<big>ואם</big> רצית לחלק המרובע שהוא שוה הצלעות אך הוא בלתי נצב הזויות כזה לשני חלקים | ||
| + | |- | ||
| + | | | ||
| + | :In the first way: divide AB into two [equal] parts at point H, then draw a [straight] line from point H, parallel to AD and BG, which is HZ, and by this the quadrangle is divided into two equal parts. | ||
| + | |style="text-align:right;"|מדרך הראשונה תחלק צלע א"ב לשני חלקים על נקדת ה' ותוציא מנקדת ה' קו נכחי לקו א"ד ולקו ב"ג והוא קו ה"ז ובזה נחלק המרובע לשני חלקים שוים | ||
| + | |- | ||
| + | | | ||
| + | :The proof of this division: | ||
| + | |style="text-align:right;"|<big>והמופת</big> על החלוקה הזו | ||
| + | |- | ||
| + | | | ||
| + | :*<math>\scriptstyle AH=HB</math> | ||
| + | |style="text-align:right;"|כי א"ה כמו ה"ב | ||
| + | |- | ||
| + | | | ||
| + | :*<math>\scriptstyle AD=BG</math> | ||
| + | |style="text-align:right;"|וא"ד כמו ב"ג | ||
| + | |- | ||
| + | | | ||
| + | :*HZ is shared by both. | ||
| + | |style="text-align:right;"|וה"ז משותף | ||
| + | |- | ||
| + | | | ||
| + | :*<math>\scriptstyle\measuredangle DAH=\measuredangle ZHB</math> | ||
| + | |style="text-align:right;"|וזוית דא"ה שוה לזוית זה"ב | ||
| + | |- | ||
| + | | | ||
| + | :*<math>\scriptstyle\measuredangle ADZ=\measuredangle HZG</math> | ||
| + | |style="text-align:right;"|וזוית אד"ז שוה לזוית הז"ג | ||
| + | |- | ||
| + | | | ||
| + | :*<math>\scriptstyle\measuredangle AHZ=\measuredangle HBG</math> | ||
| + | |style="text-align:right;"|וזוית אה"ז שוה לזוית הב"ג | ||
| + | |- | ||
| + | | | ||
| + | :*<math>\scriptstyle\measuredangle AZD=\measuredangle BGZ</math> | ||
| + | |style="text-align:right;"|וזוית אז"ד שוה לזוית בג"ז | ||
| + | |- | ||
| + | | | ||
| + | :The area of one equal to [the area of] the other. | ||
| + | |style="text-align:right;"|והשטח האחד שוה לחברו | ||
| + | |- | ||
| + | | | ||
| + | *Do the same if you want to divide it into three or four [equal] parts. | ||
| + | |style="text-align:right;"|וככה תעשה אם רצית לחלקו בג' וד' חלקים | ||
| + | |- | ||
| + | | | ||
| + | *Also, if you wish to divide it from AD in this same way and according to these same proofs. | ||
| + | |style="text-align:right;"|גם אם רצית לחלקו מצלע א"ד חלקהו בכזה הדרך בעצמו ומאלה המופתים בעצמם | ||
| + | |- | ||
| + | | | ||
| + | *If you wish to divide it through the vertices into two equal parts: | ||
| + | |style="text-align:right;"|ואם רצית לחלקו מן דרך הזויות אם רצית לחלקו בשני חלקים שוים | ||
| + | |- | ||
| + | | | ||
| + | :Draw diagonal AG, so it is divided into two equal parts. | ||
| + | |style="text-align:right;"|תגיע אלכסון א"ג ויחלק על שני חלקים שוים | ||
| + | |- | ||
| + | | | ||
| + | :The proof of this division: | ||
| + | :<math>\scriptstyle\triangle ABG=\triangle ADG</math> | ||
| + | |style="text-align:right;"|<big>והמופת</big> על החלוקה הזאת כי משולש אב"ג שוה למשולש אד"ג | ||
| + | |- | ||
| + | | | ||
| + | :*<math>\scriptstyle AB=DG</math> | ||
| + | |style="text-align:right;"|צלע א"ב כמו צלע ד"ג | ||
| + | |- | ||
| + | | | ||
| + | :*<math>\scriptstyle AD=BG</math> | ||
| + | |style="text-align:right;"|וצלע א"ד כמו צלע ב"ג | ||
| + | |- | ||
| + | | | ||
| + | :*<math>\scriptstyle\measuredangle ABG=\measuredangle ADG</math> since they are sides of the same base. | ||
| + | |style="text-align:right;"|וזוית אב"ג שוה לזוית אד"ג כי הם מיתרי תושבת אחת | ||
| + | |- | ||
| + | | | ||
| + | :Therefore the two triangles are equal and they are the two parts of the quadrilateral. | ||
| + | |style="text-align:right;"|אם כן שני המשולשים שוים והם שני חלקי המרובע | ||
| + | |- | ||
| + | | | ||
| + | :Do likewise if you wish to divide it by using diagonal BD, relying on this same proof. | ||
| + | |style="text-align:right;"|גם ככה תעשה אם רצית לחלקו מאלכסון ב"ד בכזה המופת בעצמו | ||
| + | |- | ||
| + | | | ||
| + | *If you wish to divide it into three equal parts by the first way: | ||
| + | |style="text-align:right;"|<big>ואם</big> רצית לחלקו לשלשה חלקים שוים אם מהדרך הראשונה | ||
| + | |- | ||
| + | | | ||
| + | :Divide AB into three equal parts: AH, HZ, ZB, then draw line HC parallel to AD and line ZT parallel to BG, so the quadrangle is divided into three equal parts. | ||
| + | |style="text-align:right;"|חלק צלע א"ב לג' חלקים שוים על א"ה ה"ז וז"ב והוצא קו ה"ח נכוחי לא"ד וקו ז"ט נכוחי לב"ג ויחלק המרובע לג' חלקים שוים | ||
| + | |- | ||
| + | | | ||
| + | *Likewise if you want to divide it into four parts or more. | ||
| + | |style="text-align:right;"|וכן אם רצית לחלקו לד' חלקים ויותר | ||
| + | |- | ||
| + | | | ||
| + | :The proof of this division: | ||
| + | |style="text-align:right;"|<big>והמופת</big> על החלוקה הזאת | ||
| + | |- | ||
| + | | | ||
| + | :*<math>\scriptstyle AD=HC</math> | ||
| + | |style="text-align:right;"|כי צלע א"ד שוה לצלע ה"ח | ||
| + | |- | ||
| + | | | ||
| + | :*<math>\scriptstyle HC=ZT</math> | ||
| + | |style="text-align:right;"|וצלע ה"ח שוה לצלע ז"ט | ||
| + | |- | ||
| + | | | ||
| + | :*<math>\scriptstyle ZT=BG</math> | ||
| + | |style="text-align:right;"|וצלע ז"ט שוה לצלע ב"ג | ||
| + | |- | ||
| + | | | ||
| + | :*<math>\scriptstyle AH=HZ=ZB</math> | ||
| + | |style="text-align:right;"|וצלע א"ה שוה לצלע ה"ז ז"ב | ||
| + | |- | ||
| + | | | ||
| + | :*<math>\scriptstyle\measuredangle HAD=\measuredangle ZHC=\measuredangle BZT</math> | ||
| + | |style="text-align:right;"|וזוית הא"ד שוה לזויות זה"ח בז"ט | ||
| + | |- | ||
| + | | | ||
| + | :*<math>\scriptstyle\measuredangle ADC=\measuredangle HCT=\measuredangle ZTG</math> | ||
| + | |style="text-align:right;"|וזוית אד"ח שוה לזוית הח"ט זט"ג | ||
| + | |- | ||
| + | | | ||
| + | :*<math>\scriptstyle\measuredangle HCD=\measuredangle ZTC=\measuredangle BGT</math> | ||
| + | |style="text-align:right;"|וזוית הח"ד שוה לזויות זט"ח בג"ט | ||
| + | |- | ||
| + | | | ||
| + | :Therefore the parallelograms are equal and they are three parts of the quadrangle. | ||
| + | |style="text-align:right;"|אם כן גם השטחים נכחיי הצלעות שוים והם ג' חלקי המרובע | ||
| + | |- | ||
| + | | | ||
| + | *If you wish to divide it in the second way, do as follows: | ||
| + | |style="text-align:right;"|<big>ואם</big> רצית לחלקו מדרך השנית עשה כך | ||
| + | |- | ||
| + | | | ||
| + | :Divide DG, so that from point D to point H it is two-thirds of the side, and draw AH. | ||
| + | |style="text-align:right;"|חלק צלע ד"ג ויהיה מנקדת ד' עד נקדת ה' שני שלישי הצלע ותגיע א"ה | ||
| + | |- | ||
| + | | | ||
| + | :Divide also AB, so that from point B to point Z it is two-thirds of the side, and draw GZ. | ||
| + | |style="text-align:right;"|עוד חלק צלע א"ב ויהיה מנקדת ב' עד נקדת ז' שני שלישי הצלע ותגיע ג"ז | ||
| + | |- | ||
| + | | | ||
| + | :By this the quadrangle is divided into three equal parts. | ||
| + | |style="text-align:right;"|ובזה נחלק המרובע לג' חלקים שוים | ||
| + | |- | ||
| + | | | ||
| + | :The proof of this division: | ||
| + | |style="text-align:right;"|<big>והמופת</big> על החלוקה הזאת | ||
| + | |- | ||
| + | | | ||
| + | :The ratio of <math>\scriptstyle\triangle ADH</math> to parallelogram AZHG is as half the ratio of its base to its base, since they are between two parallel lines. | ||
| + | |style="text-align:right;"|כי יחס משולש אד"ה אל שטח אזה"ג נכחי הצלעות כחצי יחס תושבתו אל תושבתו כי הם בין שני קוים נכוחיים | ||
| + | |- | ||
| + | | | ||
| + | :And so is the ratio of <math>\scriptstyle\triangle GBZ</math> to parallelogram AZHG, for the same reason. | ||
| + | |style="text-align:right;"|וכן יחס משולש גב"ז אל שטח אזה"ג לזאת הסבה בעצמה | ||
| + | |- | ||
| + | | | ||
| + | :Half the ratio of its base to its base is the same. | ||
| + | |style="text-align:right;"|וחצי יחס תושבתו על תושבתו כמוהו | ||
| + | |- | ||
| + | | | ||
| + | :Therefore, the two triangles and the [parallelogram] are three equal parts and they are the parts of the quadrangle. | ||
| + | |style="text-align:right;"|אם כן שני המשולשים והשטח שלשה חלקים שוים והם חלקי המרובע | ||
| + | |- | ||
| + | | | ||
| + | *If you wish to divide it into four equal parts: | ||
| + | |style="text-align:right;"|ואם רצית לחלקו לד' חלקי' שוים | ||
| + | |- | ||
| + | | | ||
| + | :Divide AB into two equal parts at point H. | ||
| + | |style="text-align:right;"|תחלק צלע א"ב לשני חלקים שוים על נקדת ה‫' | ||
| + | |- | ||
| + | | | ||
| + | :Divide DG similarly at point Z. | ||
| + | |style="text-align:right;"|גם ככה תחלק צלע ד"ג על נקדת ז‫' | ||
| + | |- | ||
| + | | | ||
| + | :Draw lines AZ and GH. | ||
| + | |style="text-align:right;"|ותגיע א"ז וג"ה | ||
| + | |- | ||
| + | | | ||
| + | :Divide AH also into two equal parts at point C | ||
| + | |style="text-align:right;"|עוד תחלק א"ה לשני חלקים שוים על נקודת ח‫' | ||
| + | |- | ||
| + | | | ||
| + | :Divide ZG similarly at point T. | ||
| + | |style="text-align:right;"|גם תחלק ז"ג על נקדת ט‫' | ||
| + | |- | ||
| + | | | ||
| + | :Draw CT and by this the quadrangle is divided into four equal parts. | ||
| + | |style="text-align:right;"|ותגיע ח"ט ובזה נחלק המרובע אל ארבעה ‫<ref>58v</ref>חלקים שוים | ||
| + | |- | ||
| + | | | ||
| + | :The proof of this division: | ||
| + | |style="text-align:right;"|<big>והמופת</big> על החלוקה הזאת | ||
| + | |- | ||
| + | | | ||
| + | :Since the base of <math>\scriptstyle\triangle ADZ</math> is as the base of parallelogram AHZG and they are between parallel lines, this [parallelogram] is as double of each of the triangles ADZ and GBH. | ||
| + | :<math>\scriptstyle AHZG=2\sdot\triangle ADZ=2\sdot\triangle GBH</math> | ||
| + | |style="text-align:right;"|לפי שתושבת משולש אד"ז כתושבת שטח אהז"ג נכחי הצלעות והם בין שני קוים נכחיים יהיה השטח הזה כפל כל אחד ממשולשי אד"ז גב"ה | ||
| + | |- | ||
| + | | | ||
| + | :When we divide it into two equal parts: [parallelogram] ACZT and [parallelogram] CHTZ, each of these [parallelograms] that are parts of the one [parallelogram] is equal to each of the triangles. | ||
| + | |style="text-align:right;"|וכאשר חלקנו זה לשני חלקים שוים לשטח אחז"ט ולשטח חהט"ג יהיה כל אחד מהשטחים שהם חלקי השטח האחד שוה לכל אחד מהמשולשים | ||
| + | |- | ||
| + | | | ||
| + | :The two [parallelograms] with the two triangles are all parts of the quadrangle, so the whole quadrangle is divided into four equal parts. | ||
| + | |style="text-align:right;"|ושני השטחים עם שני המשולשים הם כל חלקי המרובע הנה נחלק כל המרובע לד' חלקים שוים | ||
| + | |- | ||
| + | | | ||
| + | *If you wish to divide it into four equal parts in the way of the two diagonals: | ||
| + | |style="text-align:right;"|עוד אם רצית לחלקו לארבעה חלקים שוים ‫[מדרך שני האלכסונים | ||
| + | |- | ||
| + | | | ||
| + | :Draw AG and BD, they intersect at point H, so it is divided into four equal parts. | ||
| + | |style="text-align:right;"|תגיע א"ג וכן ב"ד ויפגשו על נקדת ה' ויחלק המרבע לד' חלקים שוים‫]‫<ref>P1031 om.</ref> | ||
| + | |- | ||
| + | | | ||
| + | :The proof of this division: | ||
| + | |style="text-align:right;"|והמופת על החלוקה הזאת | ||
| + | |- | ||
| + | | | ||
| + | :<math>\scriptstyle\triangle AHB=\triangle AHD</math> | ||
| + | |style="text-align:right;"|כי משולש אה"ב שוה למשולש אה"ד | ||
| + | |- | ||
| + | | | ||
| + | :*<math>\scriptstyle AB=AD</math> | ||
| + | |style="text-align:right;"|צלע א"ב שוה לצלע א"ד | ||
| + | |- | ||
| + | | | ||
| + | :*AH is shared by both. | ||
| + | |style="text-align:right;"|וצלע א"ה משותף | ||
| + | |- | ||
| + | | | ||
| + | :*<math>\scriptstyle\measuredangle DAH=\measuredangle HAB</math> | ||
| + | |style="text-align:right;"|וזוית דא"ה שוה לזוית הא"ב | ||
| + | |- | ||
| + | | | ||
| + | ::Because <math>\scriptstyle\measuredangle AB=AD</math> | ||
| + | |style="text-align:right;"|לפי שצלע א"ב שוה לצלע א"ד | ||
| + | |- | ||
| + | | | ||
| + | :*AG is shared by both. | ||
| + | |style="text-align:right;"|וצלע א"ג משותף | ||
| + | |- | ||
| + | | | ||
| + | :*<math>\scriptstyle BG=DG</math> | ||
| + | |style="text-align:right;"|ותושבת ב"ג שוה לתושבת ד"ג | ||
| + | |- | ||
| + | | | ||
| + | :*<math>\scriptstyle\measuredangle DAG=\measuredangle GAB</math> | ||
| + | |style="text-align:right;"|תהיה זוית דא"ג שוה לזוית גא"ב | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle\measuredangle GAD</math> and <math>\scriptstyle\measuredangle GAB</math> are <math>\scriptstyle\measuredangle DAH</math> and <math>\scriptstyle\measuredangle HAB</math> | ||
| + | |style="text-align:right;"|וזוית גא"ד וגא"ב הן זויות דא"ה והא"ב | ||
| + | |- | ||
| + | | | ||
| + | :Therefore, <math>\scriptstyle\triangle AHB=\triangle AHD</math> | ||
| + | |style="text-align:right;"|אם כן משולש אה"ב שוה למשולש אה"ד | ||
| + | |- | ||
| + | | | ||
| + | :<math>\scriptstyle\triangle AHD=\triangle GHD</math> | ||
| + | |style="text-align:right;"|ומשולש אה"ד שוה למשולש גה"ד | ||
| + | |- | ||
| + | | | ||
| + | :*<math>\scriptstyle AD=GD</math> | ||
| + | |style="text-align:right;"|לפי שצלע א"ד שוה לצלע ג"ד | ||
| + | |- | ||
| + | | | ||
| + | :*DH is shared by both. | ||
| + | |style="text-align:right;"|וצלע ד"ה משותף | ||
| + | |- | ||
| + | | | ||
| + | :*<math>\scriptstyle\measuredangle ADH=\measuredangle HDG</math> | ||
| + | |style="text-align:right;"|וזוית אד"ה שוה לזוית הד"ג | ||
| + | |- | ||
| + | | | ||
| + | ::Because AD of <math>\scriptstyle\triangle ADB</math> is equal to DG of <math>\scriptstyle\triangle DBG</math> | ||
| + | |style="text-align:right;"|לפי שצלע א"ד ממשולש אד"ב שוה לצלע ד"ג ממשולש דב"ג | ||
| + | |- | ||
| + | | | ||
| + | :*DB is shared by both. | ||
| + | |style="text-align:right;"|וצלע ד"ב משותף | ||
| + | |- | ||
| + | | | ||
| + | :*<math>\scriptstyle AB=BG</math> | ||
| + | |style="text-align:right;"|ותושבת א"ב שוה לתושבת ב"ג | ||
| + | |- | ||
| + | | | ||
| + | ::So, <math>\scriptstyle\measuredangle ADB=\measuredangle BDG</math> | ||
| + | |style="text-align:right;"|אם כן זוית אד"ב שוה לזוית בד"ג | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle\measuredangle ADB</math> and <math>\scriptstyle\measuredangle BDG</math> are <math>\scriptstyle\measuredangle ADH</math> and <math>\scriptstyle\measuredangle HDG</math> | ||
| + | |style="text-align:right;"|וזויות אד"ב ובד"ג הן זויות אד"ה והד"ג | ||
| + | |- | ||
| + | | | ||
| + | :Therefore, <math>\scriptstyle\triangle AHD=\triangle GHD</math> | ||
| + | |style="text-align:right;"|אם כן משולש אה"ד שוה למשולש גה"ד | ||
| + | |- | ||
| + | | | ||
| + | :In this same way it is clear that <math>\scriptstyle\triangle GDH=\triangle GHB</math> | ||
| + | |style="text-align:right;"|ובזה הדרך בעצמו יתבאר שמשולש גד"ה שוה למשולש גה"ב | ||
| + | |- | ||
| + | | | ||
| + | :Hence, the four triangles that are all the parts of the quadrangle are equal. | ||
| + | |style="text-align:right;"|אם כן המשולשים הד' שהם כל חלקי המרובע שוים | ||
| + | |- | ||
| + | | | ||
| + | *If you want to divide it in another way, do as follows: | ||
| + | |style="text-align:right;"|ואם רצית לחלק מדרך אחרת עשה כך | ||
| + | |- | ||
| + | | | ||
| + | *Example: we wish to divide the quadrangle ABGD into four parts. | ||
| + | |style="text-align:right;"|דמיון רצינו לחלק מרובע אבג"ד לד' חלקים | ||
| + | |- | ||
| + | | | ||
| + | :We divide AD at point H, which is half the side. | ||
| + | |style="text-align:right;"|חלקנו צלע א"ד על נקדת ה' והיא חצי הצלע | ||
| + | |- | ||
| + | | | ||
| + | :We also divide BG into two halves at point Z. | ||
| + | |style="text-align:right;"|גם חלקנו צלע ב"ג לשני חצאים על נקדת ז‫' | ||
| + | |- | ||
| + | | | ||
| + | :We also divide AB into two halves at point C. | ||
| + | |style="text-align:right;"|גם חלקנו צלע א"ב לשני חציים על נקדת ח‫' | ||
| + | |- | ||
| + | | | ||
| + | :We also divide DG into two halves at point T. | ||
| + | |style="text-align:right;"|גם חלקנו צלע ד"ג לשני חצאים על נקדת ט‫' | ||
| + | |- | ||
| + | | | ||
| + | :Then we draw HZ and CT. | ||
| + | |style="text-align:right;"|אחר כך הגענו ה"ז ח"ט | ||
| + | |- | ||
| + | | | ||
| + | :They intersect at point K, so the quadrangle is divided into four equal parts. | ||
| + | |style="text-align:right;"|ויפגשו על נקדת כ' ויחלק המרובע לד' חלקים שוים | ||
| + | |- | ||
| + | | | ||
| + | :The proof of this division: | ||
| + | |style="text-align:right;"|והמופת על החלוקה הזאת | ||
| + | |- | ||
| + | | | ||
| + | :*<math>\scriptstyle HZ\parallel DG</math> | ||
| + | |style="text-align:right;"|לפי שצלע ה"ז נכחי לצלע ד"ג | ||
| + | |- | ||
| + | | | ||
| + | ::Because the distances of their ends are equal. | ||
| + | |style="text-align:right;"|כי [מרחקי קצוותיהם]‫<ref>P1031: מרחק תושבתו של כל א' מהם</ref> שוה | ||
| + | |- | ||
| + | | | ||
| + | :*<math>\scriptstyle GT=TD</math> | ||
| + | |style="text-align:right;"|ותושבת ג"ט שוה לתושבת ט"ד | ||
| + | |- | ||
| + | | | ||
| + | :Therefore, quadrangle GZKT is equal to quadrangle TKHD. | ||
| + | |style="text-align:right;"|יהיה מרובע גזכ"ט שוה למרובע טכה"ד | ||
| + | |- | ||
| + | | | ||
| + | :*<math>\scriptstyle CT\parallel AD</math> | ||
| + | |style="text-align:right;"|ולפי שצלע ח"ט נכחי לצלע א"ד | ||
| + | |- | ||
| + | | | ||
| + | :*<math>\scriptstyle DH=HA</math> | ||
| + | |style="text-align:right;"|ותושבת ד"ה שוה לתושבת ה"א | ||
| + | |- | ||
| + | | | ||
| + | :Therefore, quadrangle GZKT is equal to quadrangle HKCA. | ||
| + | |style="text-align:right;"|יהיה מרובע טכה"ד שוה למרובע הכח"א | ||
| + | |- | ||
| + | | | ||
| + | :In this same way, quadrangle CBZK is equal to them. | ||
| + | |style="text-align:right;"|ובזה הדרך בעצמו ישוה להם מרובע חבז"כ | ||
| + | |- | ||
| + | | | ||
| + | :So, these four quadrangles are all parts of the great quadrangle and they are equal. | ||
| + | |style="text-align:right;"|ואלה ד' מרובעי' הם כל חלקי המרובע הגדול והם שוים | ||
| + | |- | ||
| + | !<span style=color:Green>Parallelogram</span> | ||
| + | | | ||
| + | |- | ||
| + | |This way of dividing the rhombus is the way of dividing the parallelogram [lit. long quadrangle that is not right-angled, but its sides are parallel] and its proofs are as its proofs. | ||
| + | |style="text-align:right;"|ודרך חלוקת זה המרובע הבלתי נצב הזויות הוא דרך חלוקת המרובע הארוך הבלתי נצב הזויות הנכחי הצלעות ומופתיו כמופתיו | ||
| + | |- | ||
| + | !<span style=color:Green>Trapezoid</span> | ||
| + | | | ||
| + | |- | ||
| + | |Now, we will explain the division of the trapezoids, since they are of many types: | ||
| + | |style="text-align:right;"|<big>ומעתה</big> נבאר חלוקת הנוטים כי הם מינים הרבה | ||
| + | |- | ||
| + | !<span style=color:Green>Isosceles Trapezoid</span> | ||
| + | | | ||
| + | |- | ||
| + | |We start with the trapezoid whose legs are equal, but its upper base and its bottom base are not equal, and its upper base is parallel to its bottom base, which is called truncated triangle, like this: | ||
| + | |style="text-align:right;"|ונחל מן הנוטה אשר צלעיו שוים וראשו ותושבתו בלתי שוים וראשו נכח תושבתו והוא אשר יקרא משולש חתוך הראש כזה | ||
| + | |- | ||
| + | |Among them there is one that is right angled, but its upper base is not parallel to its bottom base. | ||
| + | |style="text-align:right;"|ויש ממנו נצב הזויות אך אין ראשו נכח תושבתו | ||
| + | |- | ||
| + | | | ||
| + | *When we wish to divide trapezoid ABGD, whose upper base is parallel to its bottom base, and its two legs are equal, into two equal parts: | ||
| + | |style="text-align:right;"|וכאשר רצינו לחלק נוטה אבג"ד שראשו נכח תושבתו ושתי צלעותיו שוים לשני חלקים שוים | ||
| + | |- | ||
| + | | | ||
| + | :We divide AB into two equal parts at point H. | ||
| + | |style="text-align:right;"|נחלק צלע א"ב לשני חלקים שוים על נקדת ה‫' | ||
| + | |- | ||
| + | | | ||
| + | :We also divide GD at point Z. | ||
| + | |style="text-align:right;"|וכן ‫<ref>59r</ref>נחלק צלע ג"ד על נקדת ז‫' | ||
| + | |- | ||
| + | | | ||
| + | :We draw HZ, then ABGD is divided into two equal parts. | ||
| + | |style="text-align:right;"|ונגיע ה"ז ויחלק נוטה אבג"ד לשני חלקים שוים | ||
| + | |- | ||
| + | | | ||
| + | :The proof of this division: | ||
| + | |style="text-align:right;"|והמופת על החלוקה הזאת | ||
| + | |- | ||
| + | | | ||
| + | :*<math>\scriptstyle AD=BG</math> | ||
| + | |style="text-align:right;"|לפי שצלע א"ד שוה לצלע ב"ג | ||
| + | |- | ||
| + | | | ||
| + | :*<math>\scriptstyle DZ=ZG</math> | ||
| + | |style="text-align:right;"|ותושבת ד"ז לתושבת ז"ג | ||
| + | |- | ||
| + | | | ||
| + | :*<math>\scriptstyle AH=HB</math> | ||
| + | |style="text-align:right;"|וראש א"ה לראש ה"ב | ||
| + | |- | ||
| + | | | ||
| + | :*HZ is shared by both. | ||
| + | |style="text-align:right;"|וצלע ה"ז הוא משותף | ||
| + | |- | ||
| + | | | ||
| + | :Therefore trapezoid AHDZ is equal the trapezoid HBZG and they are the whole trapezoid ABGD, so it is divided into two equal parts. | ||
| + | |style="text-align:right;"|אם כן נוטה אהד"ז שוה לנוטה הבז"ג והם כל נוטה אבג"ד והנה נחלק לשני חלקים שוים | ||
| + | |- | ||
| + | | | ||
| + | *If you wish to divide it into three equal parts and the upper base is as half the bottom base: | ||
| + | |style="text-align:right;"|ואם רצית לחלקו לג' חלקים שוים והיה הראש כמו מחצית התושבת | ||
| + | |- | ||
| + | | | ||
| + | :Divide the bottom base into two equal parts at point Z. | ||
| + | |style="text-align:right;"|תחלק התושבת לשני חלקים שוים על נקדת ז‫' | ||
| + | |- | ||
| + | | | ||
| + | :Draw lines AZ and BZ, then the trapezoid is divided into three equal triangles. | ||
| + | |style="text-align:right;"|ותגיע א"ז ב"ז ויחלק הנוטה לג' משולשים שוים | ||
| + | |- | ||
| + | | | ||
| + | :The proof of this division: | ||
| + | |style="text-align:right;"|והמופת על החלוקה הזאת | ||
| + | |- | ||
| + | | | ||
| + | :ABDZ is a parallelogram and it is double <math>\scriptstyle\triangle ADZ</math>, because they have the same base and they are between two parallel lines. | ||
| + | |style="text-align:right;"|ששטח א"ב ד"ז נכחי הצלעות והוא כפל משולש אד"ז כי הם על תושבת אחת ובין שני קוים נכוחיים | ||
| + | |- | ||
| + | | | ||
| + | :<math>\scriptstyle2\sdot\triangle ADZ=\triangle ADZ+\triangle AZB</math> | ||
| + | |style="text-align:right;"|וכפל משלש אד"ז שוה לשני משולשי אד"ז אז"ב | ||
| + | |- | ||
| + | | | ||
| + | :ABGZ is double <math>\scriptstyle\triangle BZG</math>, for the same reason itself. | ||
| + | |style="text-align:right;"|עוד [שטח] אבג"ז שוה לכפל משולש בז"ג לסבה הזאת בעצמה | ||
| + | |- | ||
| + | | | ||
| + | :<math>\scriptstyle2\sdot\triangle BZG=\triangle AZB+\triangle BZG</math> | ||
| + | |style="text-align:right;"|וכפל משולש בז"ג שוה לשני משולשי אז"ב בז"ג | ||
| + | |- | ||
| + | | | ||
| + | :Therefore the three triangles are also equal, because when you subtract ABZ that is common to both, <math>\scriptstyle\triangle ADZ=\triangle BZG</math> remains and they are all the parts of the trapezoid. | ||
| + | |style="text-align:right;"|א"כ גם ג' המשלשים שוים כי כשתפיל אב"ז המשותף ישאר משלש אד"ז שוה למשלש בז"ג והם כל חלקי הנוטה | ||
| + | |- | ||
| + | | | ||
| + | *If the upper base of the trapezoid is not half the bottom base, but greater or smaller than it, and you wish to divide it into three equal parts, do as follows: | ||
| + | |style="text-align:right;"|אמנם אם ראש הנוטה איננו במחצית התושבת אבל עודף עליו ותרצה לחלק אותו בשלשה חלקים שוים או יהיה פוחת ממנו תעשה כך | ||
| + | |- | ||
| + | | | ||
| + | :Find its area, then divide the area into three parts and so is its division, i.e. a third of the area is a third of the trapezoid and so is each part. | ||
| + | |style="text-align:right;"|תמצא תשברתו אחר כך חלק מספר התשבורת לג' חלקים וככה יהיה חלוקתו כלומר מספר שלישית השברים הוא שלישית הנוטה וככה כל חלק וחלק | ||
| + | |- | ||
| + | | | ||
| + | *Divide the trapezoid that is right angled, but its upper base is not parallel to its bottom base, like this. | ||
| + | |style="text-align:right;"|וככה תחלק את הנוטה שהוא נצב הזויות ואין ראשו נכח תושבתו | ||
| + | |- | ||
| + | !<span style=color:Green>Circle</span> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | *Also, if you wish to divide the circle into two parts: | ||
| + | |style="text-align:right;"|גם אם רצית לחלק העגולה לשני חלקים שוים | ||
| + | |- | ||
| + | | | ||
| + | :Draw the diameter of the circle and when it passes the center it is divided into two equal parts. | ||
| + | |style="text-align:right;"|תוציא אלכסון העגולה ובעברה על המרכז תחלק לשני חלקים שוים | ||
| + | |- | ||
| + | | | ||
| + | :The proof of this division is that the arc of half the circle is equal to the arc of its other half and the diameter is common to both, so one shape is equal to the other. | ||
| + | |style="text-align:right;"|והמופת על החלוקה הזאת כי קשת חצי העגולה שוה לקשת חציה האחר והאלכסון משותף אם כן התמונה האחת שוה לאחרת | ||
| + | |- | ||
| + | | | ||
| + | *If you wish to divide it into three equal parts of more, as many as you wish: | ||
| + | |style="text-align:right;"|ואם רצית לחלקה לג' חלקים שוים או ליותר כמה חלקים שתרצה | ||
| + | |- | ||
| + | | | ||
| + | :Divide the [perimeter] as the number of parts you want and mark points as the number of the parts of the [perimeter]. Then, draw a straight line from each point to the center, so the circle is divided into the number of parts you wanted. | ||
| + | |style="text-align:right;"|תחלק העגולה כמספר החלקי' שתרצה ותסמן נקדות כמספר החלקים על העגולה אח"כ הוצא מכל נקודה קו ישר אל המרכז והנה נחלקה העגולה על מספר החלקים שרצית | ||
| + | |- | ||
| + | | | ||
| + | :The proof of this division: the arcs are equal and the lines are equal, because they extend from the perimeter to the center, so the circle is divided into equal triangles, whose bases are equal arcs of the circle. | ||
| + | |style="text-align:right;"|והמופת על החלוקה הזאת כי הקשתות שוות והקוים שוים כי הם יוצאים מהמקיף אל המרכז ואם כן נחלקה העגולה אל משלשים שוים אשר תושבותם קשתות שוות מהעגולה | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | === The Third Section on the Measuring of Solids === | ||
| + | |||
| + | |style="text-align:right;"|<big>החלק השלישי</big> במדידת הגופנים | ||
| + | |- | ||
| + | | | ||
| + | ==== The First Chapter ==== | ||
| + | |||
| + | |style="width:45%; text-align:right;"|<big>הפרק הראשון</big> | ||
| + | |- | ||
| + | |Know that the solids are those that have length, width and height. | ||
| + | |style="text-align:right;"|דע שהגופנים הם אשר יש להם אורך ורחב וגובה | ||
| + | |- | ||
| + | | | ||
| + | *Among them are those that are called pyramid, of which all the bases are triangular [= triangular pyramid], like this: | ||
| + | |style="text-align:right;"|ויש מהם אשר יקרא מחודד וכל תושבותיו משולשים כזה | ||
| + | |- | ||
| + | | | ||
| + | :It is a shape that rises in height and ends at one point | ||
| + | |style="text-align:right;"|והיא תמונה אשר תעלה בגובהה ותכלה אל נקדה אחת | ||
| + | |- | ||
| + | | | ||
| + | *Among them are those, whose base is quadrilateral and their faces are triangular [= quadrilateral pyramid], like this: | ||
| + | |style="text-align:right;"|ויש מהם אשר תושבתו מרובע וצדדיו משולשים כזה | ||
| + | |- | ||
| + | | | ||
| + | :They rise in height to one point. | ||
| + | |style="text-align:right;"|ויפלו בגבהם אל נקדה | ||
| + | |- | ||
| + | | | ||
| + | *Among them are those, whose base is circular, that rise in height and end at one point [= cone], like this: | ||
| + | |style="text-align:right;"|ויש מהם שתושבתם עולה בשטח עגול ויכלה בגבהו אל נקדה כזה | ||
| + | |- | ||
| + | | | ||
| + | *Among them are those, whose base is a polygon, that end at one point [= polygonal pyramid]. | ||
| + | |style="text-align:right;"|‫<ref>59v</ref>ויש מהם שתושבתם רבת הזויות ותכלה אל נקדה | ||
| + | |- | ||
| + | | | ||
| + | *Among them are those, whose bottom base is a triangle, their upper base is a triangle, their faces are quadrangles that rise laterally at right angle [= triangular prism], like this: | ||
| + | |style="text-align:right;"|ויש מהם שתושבתם משולש וראשם משלש וצדדיהם המרובעים עולים על צד זוית נצבת כזה | ||
| + | |- | ||
| + | | | ||
| + | *[Among them] are those that rise to a point, but end before reaching the point [= truncated pyramid], like this: | ||
| + | |style="text-align:right;"|ויש שאינם עולים על נקדה אבל נפסקים קודם שיגיעו אל הנקדה כזה | ||
| + | |- | ||
| + | | | ||
| + | *Among them is the shape of a sphere, like this: | ||
| + | |style="text-align:right;"|ויש מהם תמונת הכדור כזה | ||
| + | |- | ||
| + | |We begin to explain the measurement of the shape, whose bottom base is quadrangular, its upper base is quadrangular, and its faces are quadrangular, rising at right angle. | ||
| + | |style="text-align:right;"|<big>ונחל</big> לפרש מדידת התמונה שתושבתה מרובע וראש המרובע וצדדיה מרבעים עולים על זויות נצבות | ||
| + | |- | ||
| + | !<span style=color:Green>Cube</span> | ||
| + | | | ||
| + | |- | ||
| + | |I say that if the length, width and the height of this shape are equal, its measurement is as follows: | ||
| + | |style="text-align:right;"|ואומר שזאת התמונה אם היא שוה ארכה ורחבה וגבהה מדידתה כן | ||
| + | |- | ||
| + | |We multiply its length by its width, then we multiply the product by its height and this is the volume of this shape that is called a cube. | ||
| + | |style="text-align:right;"|נכפול ארכה על רחבה וההוה נכפלהו על גבהה וככה מדידת זאת התמונה והיא הנקראת מעוקב | ||
| + | |- | ||
| + | | | ||
| + | *Example: its length is ten, its altitude is ten, and its breadth is ten. | ||
| + | |style="text-align:right;"|דמיון ארכה עשרה גבהה עשרה ורחבה עשרה | ||
| + | |- | ||
| + | | | ||
| + | :We multiply 10 by 10; it is 100. We multiply also 10 by 100; it is 1000 and this is the volume of this shape. | ||
| + | :<math>\scriptstyle{\color{blue}{10\sdot10\sdot10=10\sdot100=1000}}</math> | ||
| + | |style="text-align:right;"|כפלנו י' על י' ההוה ק' עוד כפלנו י' על ק' ההוה אלף והוא מדידת זאת התמונה | ||
| + | |- | ||
| + | !<span style=color:Green>Cuboid</span> | ||
| + | | | ||
| + | |- | ||
| + | |If one of the three dimensions is greater than the others, or each is different from the other, we measure it also this way: | ||
| + | |style="text-align:right;"|ואם הצד הא' מן הג' יותר מהאחרים או כל אחד לא ישוה לחברו גם כן ככה נמדדהו | ||
| + | |- | ||
| + | | | ||
| + | *Example: the length is 10, the breadth is 15, and the altitude is 20. | ||
| + | |style="text-align:right;"|דמיון האורך י' והרחב ט"ו והגובה כ‫' | ||
| + | |- | ||
| + | | | ||
| + | :We multiply 10 by 15; it is 150. We multiply it by 20; it is 3000 and this is its volume. | ||
| + | :<math>\scriptstyle{\color{blue}{10\sdot15\sdot20=150\sdot20=3000}}</math> | ||
| + | |style="text-align:right;"|נכפול י' על ט"ו ההוה ק"נ נכפלם על כ' ההוה ג' אלפים וזאת היא מדידתה | ||
| + | |- | ||
| + | |Know that the cubit, by which we measure the solid shapes is cubit in length, cubit in width, and cubit in height, and it is called solid cubit. | ||
| + | |style="text-align:right;"|ודע שהאמה אשר בה אנו מודדי' התמונות הגופניות היא אמה אורך ואמה רחב ואמה גובה וזאת תקרא אמה מוגשמת | ||
| + | |- | ||
| + | !<span style=color:Green>Space diagonal</span> | ||
| + | | | ||
| + | |- | ||
| + | |The space diagonal of these shapes is drawn from one vertex at its upper base to the opposite vertex at its bottom base. | ||
| + | |style="text-align:right;"|ואלכסון אלה התמונות יוצא מזויות אחת אשר בראשה אל נכח הזוית אשר בתושבתה | ||
| + | |- | ||
| + | | | ||
| + | :For instance, if it is drawn from the northeastern vertex at its upper base, it reaches to the southwestern vertex at its bottom base. | ||
| + | |style="text-align:right;"|דמיון אם יצא בראשה מזויות מזרחי' צפונית יגיע בתושבתה אל זוית מערבית דרומית | ||
| + | |- | ||
| + | |The measurement of the length of the space diagonal is as follows: | ||
| + | |style="text-align:right;"|ומדידת אורך האלכסון כך | ||
| + | |- | ||
| + | | | ||
| + | *Example: a cube shape, each side of which is 10. | ||
| + | |style="text-align:right;"|דמיון בצורת המעוקב אשר כל צלע מצלעותיו י‫' | ||
| + | |- | ||
| + | | | ||
| + | :We take the square of the diagonal of the base, add it to the square of the altitude, and extract its root and it is the length of the required space diagonal. | ||
| + | |style="text-align:right;"|נקח מרובע אלכסון התושבת ונחברהו עם מרובע הגובה ונקח שרשו והוא אורך האלכסון המבוקש | ||
| + | |- | ||
| + | | | ||
| + | ::It is known that the square of the diagonal of the base is 200. We add it to the square of the altitude, which is 100; the result is 300. We extract its root; it is approximately 16, a quarter, and one part of 15. | ||
| + | ::<math>\scriptstyle{\color{blue}{\sqrt{\sqrt{10^2+10^2}^2+10^2}=\sqrt{200+100}=\sqrt{300}\approx16+\frac{1}{4}+\frac{1}{15}}}</math> | ||
| + | |style="text-align:right;"|וידוע שמרובע אלכסון התושבת הוא ר' ונחברהו עם מרובע הגובה שהוא ק' עלו ש' ונקח שרשם והם י"ו ורביע וחלק א' מט"ו בקרוב | ||
| + | |- | ||
| + | |We measure the space diagonal of the shapes whose length is greater than their breadth [= cuboids] also this way: by that we find the diagonal of the base, add its square to the square of the altitude, then extract the root and this is the length of the space diagonal of the shape. | ||
| + | |style="text-align:right;"|גם ככה נמדוד אלכסון התמונות אשר ארכם יותר מרחבם כשנמצא אלכסון התושבת ונחבר מרובעו עם מרובע הגובה ונקח השרש והוא אורך אלכסון התמונה | ||
| + | |- | ||
| + | !<span style=color:Green>diagonal of the base</span> | ||
| + | | | ||
| + | |- | ||
| + | |When we want to find the diagonal of the base, we add the square of the long side of the base to the square of its short side, then extract the root and it is the diagonal of the base. | ||
| + | |style="text-align:right;"|וכאשר נרצה למצוא אלכסון התושבת נחבר מרובע צלע התושבת הארוך עם מרובע צלעו הקצר ונקח השרש והוא ארך אלכסון התושבת | ||
| + | |- | ||
| + | !<span style=color:Green>Prism</span> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | *If the [bases] of the shape are not at right angles, but they are as a parallelogram or rhombus, measure its base, as you know the measuring of these shapes, then multiply the result by the altitude and this is the volume of such shapes. | ||
| + | |style="text-align:right;"|ואם היתה התמונה צדדיה בלתי עולים על זויות נצבות אבל דומים למעויין או תהיינה מעויינות תמדוד תושבתה כמו שידעת במדידת הצורות האלה אחר כך מה שיצא כפלהו על הגובה והוא מדידת זאת התמונה כאלה | ||
| + | |- | ||
| + | | | ||
| + | *If its bottom base is triangular, its upper base is triangular, and its faces are quadrangular, find the area of the triangle that is its base, multiply it by the altitude and the result is the volume of this shape, provided that the triangle of its upper base and the triangle of its bottom base are equal. | ||
| + | |style="text-align:right;"|ואם היתה תושבתה משולש וראשה משולש וצדדיה מרובעים תמצא תשבורת המשולש אשר הוא תושבתה ותכפול אותו על הגובה והעולה היא מדידת זאת התמונה בלבד שיהיה משלש ראשה עם משולש תושבתה שוים | ||
| + | |- | ||
| + | | | ||
| + | *Similarly, if the bottom base of the shape and it upper base are equal pentagons, or equal hexagons, or others like these, find the area of the base, multiply it by the altitude and the result is [the volume of] the required shape. | ||
| + | |style="text-align:right;"|גם אם היתה תושבת התמונה וראשה מחומשים שוים או משושים שוים ויתר מאלה תמצא תשבורת ‫<ref>60r</ref>התושבת וכפלהו על הגובה והעולה היא התמונה המבוקשת | ||
| + | |- | ||
| + | | | ||
| + | *If the bottom base of the shape is a circle and the upper base is a circle equal to it, find the area of the circle and multiply it by the altitude; the result is the volume of this shape. | ||
| + | |style="text-align:right;"|ואם היתה תושבת התמונה עגולה וראש עגולה שוה לה תמצא תשבורת העגולה ותכפלהו על הגובה והעולה הוא מדידת התמונה ההיא | ||
| + | |- | ||
| + | !<span style=color:Green>Pyramid</span> | ||
| + | | | ||
| + | |- | ||
| + | |But, if the shape rises gradually and ends at one point: | ||
| + | |style="text-align:right;"|אך אם התמונה עולה בהדרגה וכלה אל נקדה | ||
| + | |- | ||
| + | | | ||
| + | *If its base is triangular, find the area of the base, multiply it by a third of the altitude and the result is the volume of this shape. | ||
| + | |style="text-align:right;"|אם תושבתה משולש תמצא תשבורת התושבת וכפלהו על שלישית הגובה והעולה הוא מדידת זאת התמונה | ||
| + | |- | ||
| + | | | ||
| + | *Likewise, if its base is quadrangular, or pentagonal, and their like, always multiply the area of the base by a third of the altitude, or the whole altitude by a third of the area of the base, and the result is the required. | ||
| + | |style="text-align:right;"|וככה אם תושבתה מרובעת או מחומשת וכדומה להם שתכפול לעולם תשבורת התושבת עם שליש הגובה או כל הגובה עם שליש תשבורת התושבת והעולה הוא המבוקש | ||
| + | |- | ||
| + | !<span style=color:Green>Cone</span> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | *Also if the base is circular, find the area of the circle, multiply it by a third of the altitude, or multiply the length of the altitude by a third of the area of the base, and the result is the required. | ||
| + | |style="text-align:right;"|וכן אם תושבתה עגולה תמצא תשבורת העגולה וכפלה על שלישית הגובה או על כפל אורך הגובה על שלישית התשבורת של התושבת והעולה הוא המבוקש | ||
| + | |- | ||
| + | !<span style=color:Green>Altitude</span> | ||
| + | | | ||
| + | |- | ||
| + | |To know how we find the altitude of the shape, I shall tell you this matter here: | ||
| + | |style="text-align:right;"|<big>ולדעת</big> איך נמצא גובה התמונה אודיעך ענינו פה | ||
| + | |- | ||
| + | |Know that the altitude is the perpendicular that is drawn at right angles from the bottom base of the shape to its top. | ||
| + | |style="text-align:right;"|<big>דע</big> שהגובה הוא עמוד יוצא מתושבת התמונה אל ראשה על זויות נצבות | ||
| + | |- | ||
| + | | | ||
| + | *If the base of the shape is a circle: | ||
| + | |style="text-align:right;"|ואם היתה תושבת התמונה עגולה | ||
| + | |- | ||
| + | | | ||
| + | :We take half of its diameter and multiply it by itself. We take the height of the lateral face and multiply it by itself. We subtract the square of half its diameter from the square of [the height of] the lateral face. We extract the root of the remainder and this is the length of the altitude. | ||
| + | |style="text-align:right;"|נקח חצי קטרה ונכפלה על עצמה עוד נקח גובה הצד ונכפלהו על עצמו ונוציא מרובע חצי קטרה ממרובע הצד והנשאר נקח גדרו והוא אורך הגובה | ||
| + | |- | ||
| + | | | ||
| + | :*Example: a circle; half its diameter is 6 and its square is 36; the height of the lateral face is 10 and its square is 100. | ||
| + | |style="text-align:right;"|דמיון עגולה חצי קטרה ו' ומרובעו ל"ו וגובה הצד י' ומרובעו ק‫' | ||
| + | |- | ||
| + | | | ||
| + | ::We subtract 36 from 100; 64 remains. We extract its root; it is 8 and this is the length of the altitude. | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{10^2-6^2}=\sqrt{100-36}=\sqrt{64}=8}}</math> | ||
| + | |style="text-align:right;"|גרענו ל"ו מן ק' ונשארו ס"ד לקחנו שרשם והם ח' וככה אורך הגובה | ||
| + | |- | ||
| + | | | ||
| + | *If the base of the shape is an equilateral triangle: | ||
| + | |style="text-align:right;"|ואם היתה התושבת של התמונה משלש שוה הצלעות | ||
| + | |- | ||
| + | | | ||
| + | :Draw a height from the top of the triangle of the base to its base and divide it into two [equal] parts. The place where it is divided is the middle of the height; mark a point there. | ||
| + | |style="text-align:right;"|תוציא עמוד מראש משלש התושבת אל תושבתו ותחלקהו לשני חלקי' ובמקום שיתחלק שם הוא מחצית העמוד וסמן שם נקדה | ||
| + | |- | ||
| + | | | ||
| + | :Divide the side of the triangle of the base also into two equal parts. The place where it is divided is the middle of the side; mark a point there. | ||
| + | |style="text-align:right;"|עוד תחלק צלע משלש התושבת לשני חלקים שוים ובמקום שיתחלק הוא מחצית הצלע וסמן שם נקדה | ||
| + | |- | ||
| + | | | ||
| + | :Then, see [the length] between the midpoint of the height and the midpoint of the side, take its square and subtract it from the square of the height of the face of the shape. Take the root of the remainder and it is the altitude of the shape. | ||
| + | |style="text-align:right;"|אח"כ ראה מה בין נקדת מחצית העמוד לנקדת מחצית הצלע וקח מרובעו וגרעהו ממרובע גובה צד התמונה וקח שרש הנשאר והוא גובה התמונה | ||
| + | |- | ||
| + | | | ||
| + | *If the base of the shape is a regular pentagon, hexagon, polygon, or a square: | ||
| + | |style="text-align:right;"|אך אם תושבת התמונה הוא מחומש שוה הצלעות והזויות או משושה והכלל רב הזויות וכן מרובע שוה הצלעות | ||
| + | |- | ||
| + | | | ||
| + | :Take half the sum of all the sides [= half the circumference] and divide the area of the base by it, square the quotient [= the apothem] and subtract its square from the square of the height of the face. Take the root of the remainder and it is the altitude of that shape. | ||
| + | |style="text-align:right;"|והוא שתקח מחצית כל הצלעות ותקבצם יחד ותחלק על המחצית ההוא מרבע התושבת והיוצא מן החלוקה תרבעהו ותוציא את מרובעו ממרובע גובה הצד והנשאר בידך קח גדרו והוא גובה התמונה ההיא | ||
| + | |- | ||
| + | !<span style=color:Green>Pyramid with irregular polygonal base</span> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | *If the sides and angles of the base are unequal, you should find the height with an instrument, then find the area of all the different triangles into which the base can be divided, by drawing [straight] lines from its vertices to its center. Sum them and multiply [the sum] by a third of the altitude. The result is the required. | ||
| + | |style="text-align:right;"|ואם לא היו הצלעות שוות כלומר צלעות התושבת גם לא הזויות אתה צריך למצוא גובה על יד כלי מעשה ואז תמצא תשבורת כל המשלשים הבלתי שוים אשר התושבת נחלקת עליהם בהוציאך קוים מזויותיה אל מרכזה ותחברם ותכפול על שלישית הגובה וההוה הוא המבוקש | ||
| + | |- | ||
| + | !<span style=color:Green>Truncated pyramid</span> | ||
| + | | | ||
| + | |- | ||
| + | |However, if the shape does not end at one point, but rather ends at a triangular or square surface, or other than these shapes, provided that its upper base is smaller than the bottom base, there are other methods [for calculating its volume], which will be explained here: | ||
| + | |style="text-align:right;"|אך אם התמונה אינה כלה אל נקדה אבל כלה אל שטח משלש או מרובע או זולת אלה בתמונה אשר תושבתה לבד שהראש קטן מן התושבת יש לה דרכים אחרי' יתבארו פה | ||
| + | |- | ||
| + | |Such shape is called a truncated pyramid, because, if you extend its faces upwards it becomes a pyramid, and we have already explained to you the rules of the pyramid. | ||
| + | |style="text-align:right;"|וזאת התמונה תקרא חתוכת הראש לפי שאם תוציא צדדיה למעלה תכלה אל מחודד וכבר בארנו לך משפטי המחודד | ||
| + | |- | ||
| + | |This shape is divided into two pyramids: the large pyramid that ends at one point, and the smaller pyramid, the base of which is the upper base of the truncated pyramid, and it rises to one point. | ||
| + | |style="text-align:right;"|והנה זאת התמונה תחלק אל שני מחודדים אל המחדד הגדול אשר כלה אל נקדה ואל ‫<ref>60v</ref>המחדד הקטן שראש התמונה חתוכת הראש תושבת לו ועולה אל הנקודה | ||
| + | |- | ||
| + | | | ||
| + | *If the bottom base is quadrangular and so is the upper base, extend the lateral sides up to the top point, then you can measure the large pyramid as you know. | ||
| + | |style="text-align:right;"|ואם היתה תושבת בעלת ד' צלעות וככה הראש תוציא אורך הצדדים עד הנקודה למעלה ואתה יכול למדוד המחודד הגדול כמו שידעת | ||
| + | |- | ||
| + | | | ||
| + | :Measure also the smaller pyramid and subtract it from the larger. The remainder is the volume of the truncated pyramid. | ||
| + | |style="text-align:right;"|עוד תמדוד המחודד הקטן וגרעהו מהגדול והנשאר הוא מדידת התמונה חתוכת הראש | ||
| + | |- | ||
| + | | | ||
| + | :Therefore, you should be taught how to find the lateral sides from the top of the shape outside it to the point: | ||
| + | |style="text-align:right;"|ולכן צריך להודיעך איך תמצא ארך הצדדים אשר הם מראש התמונה מחוץ עד הנקודה | ||
| + | |- | ||
| + | | | ||
| + | :*Example: a truncated pyramid ABGD; its bottom base is line AB, which is 6 cubits; its upper base is GD, which is 4 cubits; and its altitude in the middle, which is line HZ, is 10 cubits. | ||
| + | |style="text-align:right;"|דמיון תמונת אבג"ד חתוכת הראש ותושבתה קו א"ב והוא ו' אמות וראשה ג"ד והוא ד' אמות וגובה העמוד אשר באמצע והוא קו ה"ז י' אמות | ||
| + | |- | ||
| + | | | ||
| + | ::Multiply the upper base by the altitude; the result is 40 cubits. | ||
| + | |style="text-align:right;"|כפול הראש לכל הגובה יעלה מ' אמות | ||
| + | |- | ||
| + | | | ||
| + | ::Divide them by 2, which is the excess of the bottom base over the upper base; it is 20 and this is the length of line HT that remains from the altitude. | ||
| + | ::<math>\scriptstyle{\color{blue}{HT=\frac{GD\sdot HZ}{AB-GD}=\frac{40}{2}=20}}</math> | ||
| + | |style="text-align:right;"|חלק אותם על ב' אשר הם יתרון התושבת על הראש יהיו כ' והוא אורך קו ה"ט הנשאר מהגובה | ||
| + | |- | ||
| + | | | ||
| + | :*You want to find the length of the lateral sides that are above the upper base up to the apex: | ||
| + | |style="text-align:right;"|רצית למצוא ארך הצדדים אשר הם למעלה מהראש עד הנקודה | ||
| + | |- | ||
| + | | | ||
| + | ::Take the square of 20, which is the lenght of HT; it is 400. | ||
| + | |style="text-align:right;"|תקח מרובע כ' שהם ארך ה"ט והם ת‫' | ||
| + | |- | ||
| + | | | ||
| + | ::And the square of 2, which is the length of GH; it is 4. | ||
| + | |style="text-align:right;"|ומרובע ב' שהם אורך ג"ה והם ד‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Add it to 400; it is 404. [Take] its root, which is approximately 20 cubits and a tenth of a cubit, and it is the length of side GT that is outside the [truncated pyramid]. | ||
| + | |style="text-align:right;"|וחברם עם ת' והם ת"ד גם גדרם שהם כ' אמות ועשירית אמה בקרוב והם אורך צלע ג"ט שהוא מחוץ | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | ::<math>\scriptstyle{\color{blue}{GT=\sqrt{HT^2+GH^2}=\sqrt{20^2+2^2}=\sqrt{400+4}=\sqrt{404}\approx20+\frac{1}{10}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :*If you want to find the length of the whole lateral side of the pyramid, which is side AT: | ||
| + | |style="text-align:right;"|<big>ואם</big> רצית למצוא אורך כל צלע המחודד שהוא צלע א"ט | ||
| + | |- | ||
| + | | | ||
| + | ::Take the square of line ZT, whose lenght is 30; its square is 900. | ||
| + | |style="text-align:right;"|תקח מרובע קו ז"ט שארכו ל' ומרובעו תת"ק | ||
| + | |- | ||
| + | | | ||
| + | ::Also the square of half the bottom base that is line AZ, which is 3 cubits; its square is 9. | ||
| + | |style="text-align:right;"|גם מרובע חצי התושבת שהוא קו א"ז והוא ג' אמות ומרובעו ט‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Add it to 900; the result is 909. Extract its root, which is [approximately] 30, a tenth, and one part of 30, and it is the length of the whole lateral side. | ||
| + | |style="text-align:right;"|חברם עם תת"ק יעלו תתק"ט קח גדרם והוא ל' ועשירית א' וחלק אחד מל' והוא אורך כל התשבורת של הצלע | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | ::<math>\scriptstyle{\color{blue}{AT=\sqrt{ZT^2+AZ^2}=\sqrt{30^2+3^2}=\sqrt{900+9}=\sqrt{909}\approx30+\frac{1}{10}+\frac{1}{30}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :*Find the area of the base of the great pyramid, which is 6 by 6 cubits; the result is 36. | ||
| + | |style="text-align:right;"|תמצא תשבורת תושבת המחודד הגדול שהוא ו' על ו' אמות וההוה ל"ו | ||
| + | |- | ||
| + | | | ||
| + | ::Multiply it by a third of the altitude, which is 10, because the whole altitude is 30; the result is 360 and it is the volume of the great pyramid. | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(6\sdot6\right)\sdot\left(\frac{1}{3}\sdot30\right)=36\sdot10=360}}</math> | ||
| + | |style="text-align:right;"|כפלם עם שלישית הגובה שהם י' כי כל הגובה הם ל' וההוה ש"ס והוא מדידת המחודד הגדול | ||
| + | |- | ||
| + | | | ||
| + | ::Measure also the small pyramid, whose base is 4 by 4; its area is 16. | ||
| + | |style="text-align:right;"|עוד תשוב למדוד המחודד הקטן שתושבתו ד' על ד' ותשברתה הם י"ו | ||
| + | |- | ||
| + | | | ||
| + | ::Multiply it by 6 and 2-thirds, which is a third of its altitude; the result is 106 and 2-thirds. | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(4\sdot4\right)\sdot\left(\frac{1}{3}\sdot20\right)=16\sdot\left(6+\frac{2}{3}\right)=106+\frac{2}{3}}}</math> | ||
| + | |style="text-align:right;"|כפלם עם ו' וב' שלישיות שהם שליש גבהו וההוה ק"ו וב' שלישיות | ||
| + | |- | ||
| + | | | ||
| + | ::Subtract it from 360, which is the volume of the great pyramid; 253 and one-third remain and it is the volume of the required truncated pyramid. | ||
| + | ::<math>\scriptstyle{\color{blue}{360-\left(106+\frac{2}{3}\right)=253+\frac{1}{3}}}</math> | ||
| + | |style="text-align:right;"|תגרעם מן ש"ס שהם מדידת המחודד הגדול ישארו רנ"ג ושלישית אחד והוא מדידת התמונה חתוכת הראש המבוקשת | ||
| + | |- | ||
| + | | | ||
| + | :*Yet, since the calculation is a bit difficult, the arithmeticians introduce another shorter method: | ||
| + | |style="text-align:right;"|אך מפני שהחשבון קשה מעט נתנו בעלי החשבון דרך אחרת קצרה מזאת | ||
| + | |- | ||
| + | | | ||
| + | ::Take the square of the bottom base, which is 36, and the square of the upper base, which is 16. Multiply the root of the upper base by the root of the bottom base; the result is 24. Sum up the 36, the 16, and the 24; the result is 76. Multiply it by a third of the altitude, which is 3 and one-third; the result is 223 and one-third and this is the volume of the required truncated pyramid. | ||
| + | |style="text-align:right;"|והוא שתקח מרובע התושבת והוא ל"ו ומרובע הראש והוא י"ו ותכפול גדר הראש בגדר התושבת וההוה כ"ד תחבר הל"ו והי"ו והכ"ד והעולה ע"ו כפלם עם שלישית הגובה שהם ג' ושליש ההוה רכ"ג ושליש והוא מדידת התמונה חתוכת הראש | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | ::<math>\scriptstyle{\color{blue}{\left[6^2+4^2+\left(6\sdot4\right)\right]\sdot\left(\frac{1}{3}\sdot10\right)=\left(36+16+24\right)\sdot\left(3+\frac{1}{3}\right)=76\sdot\left(3+\frac{1}{3}\right)=253+\frac{1}{3}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | *If the bottom base of the shape is a circle and so is its upper base. | ||
| + | |style="text-align:right;"|ואם היתה תושבת התמונה עגולה וכן ראשה | ||
| + | |- | ||
| + | | | ||
| + | :*As the shape whose bottom base is a circle and the diameter of the circle is 4 cubits, the upper base is a circle, whose diameter is 2 cubits, [and the altitude is 12 cubits]. | ||
| + | |style="text-align:right;"|כגון תמונה אשר תושבתה עגולה וקטר העגולה ד' אמות וראש העגולה וקטרה ב' אמות | ||
| + | |- | ||
| + | | | ||
| + | ::The square of 4, which is the diameter of the bottom base is 16; the square of 2, which is the diameter of the upper base is 4. Multiply 2 by 4; it is 8. The total sum is 28. | ||
| + | ::<math>\scriptstyle{\color{blue}{4^2+2^2+\left(4\sdot2\right)=16+4+8=28}}</math> | ||
| + | |style="text-align:right;"|המרובע ד' אשר הוא קטר התושבת והוא י"ו ומרובע ב' שהוא קטר הראש והוא ד' וכפול ב' בד' הרי ח' והכל כ"ח | ||
| + | |- | ||
| + | | | ||
| + | ::Subtract its seventh and one part of 14; 22 remains. Multiply it by 4, which is a third of the altitude; the result is 88 and it is the volume of this shape. | ||
| + | |style="text-align:right;"|גרע [שביעיתם]‫<ref>P1031 רביעיתם</ref> וחלק אחד מי"ד נשארו כ"ב כפלם עם ד' שהוא שלישית הגובה יעלו פ"ח והוא מדידת התמונה הזאת | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | ::<math>\scriptstyle{\color{blue}{\left[\left[4^2+2^2+\left(4\sdot2\right)\right]-\left[\left[4^2+2^2+\left(4\sdot2\right)\right]\sdot\left(\frac{1}{7}+\frac{1}{14}\right)\right]\right]\sdot\left(\frac{1}{3}\sdot12\right)=\left[28-\left[28\sdot\left(\frac{1}{7}+\frac{1}{14}\right)\right]\right]\sdot4=22\sdot4=88}}</math> | ||
| + | |- | ||
| + | | | ||
| + | *Sometimes a container is found that consists of two shapes as the mentioned shape, the two bases of which are small circles and the middle it is wider. | ||
| + | |style="text-align:right;"|ולפעמים ימצא כלי אחד מחובר משתי תמונות כזאת התמונה הנזכרת שיהיו שני ראשיו עגולות קטנות ואמצעיתו רחב כזאת התמונה הנזכרת | ||
| + | |- | ||
| + | | | ||
| + | :Measure each of the two parts of the container by itself as you know, then sum them up and this is the volume of the container. | ||
| + | |style="text-align:right;"|ותמדוד כל אחת מן חלקי הכלי השנים בפני עצמו כמו שידעת וחבר שניהם וככה מדידת הכלי | ||
| + | |- | ||
| + | | | ||
| + | *If the bottom and the upper bases of the shape are equilateral triangles, but the sides of the bottom base are longer than the sides of the upper base: | ||
| + | |style="text-align:right;"|ואם היה תושבת התמונה וראשה ‫<ref>61r</ref>משלשים שוי הצלעות אך צלעות התושבת גדולות מצלעות הראש | ||
| + | |- | ||
| + | | | ||
| + | :*As the shape whose bottom base is an equilateral triangle, each of its sides are 8 cubits, upper base is an equilateral triangle, each of its sides are 4 cubits, and its altitude is 6 cubits. | ||
| + | |style="text-align:right;"|כגון תמונה שתושבתה משלש שוה הצלעו' כל אחת מצלעותיו [ח' אמות וראשה משלש שוה הצלעות כל אחת מצלעותיו]‫<ref>P1031 om.</ref> ד' אמות וגבהו ו' אמות | ||
| + | |- | ||
| + | | | ||
| + | ::You know that the area of the triangle of the bottom base is approximately 28 minus 2-sevenths and the root of this number is 5 cubits and 2-sevenths. | ||
| + | ::<math>\scriptstyle{\color{blue}{\sqrt{28-\frac{2}{7}}=5+\frac{2}{7}}}</math> | ||
| + | |style="text-align:right;"|אתה יודע כי תשבורת משלש התושבת כ"ח פחות ב' שביעיו' האחד בקירוב וגדר המספר הזה ה' אמות וב' שביעיות | ||
| + | |- | ||
| + | | | ||
| + | ::You can know the [the area of the upper base] by taking a quarter of the [bottom triangle]. | ||
| + | |style="text-align:right;"|ואתה יכול לדעת הרביע מחלק רבוע המשלשים | ||
| + | |- | ||
| + | | | ||
| + | ::You find the [area] of the triangle of the upper base is 7 minus a half of a seventh and the root of this number is two cubits, 2-sevenths and a half of a seventh. | ||
| + | ::<math>\scriptstyle{\color{blue}{\sqrt{7-\left(\frac{1}{2}\sdot\frac{1}{7}\right)}=2+\frac{4}{7}+\left(\frac{1}{2}\sdot\frac{1}{7}\right)}}</math> | ||
| + | |style="text-align:right;"|ותמצא רבוע משלש הראש ז' אמות פחות חצי שביעית וגדר המספר הזה שנים וד' שביעיות אמה וחצי שביעית | ||
| + | |- | ||
| + | | | ||
| + | ::Sum up the areas of the two triangles; it is 34 cubits and 9 parts of 14 of a cubit. | ||
| + | |style="text-align:right;"|חבר תשבורת שני המשלשים ויהיו ל"ד אמות וט' חלקים מי"ד באמה | ||
| + | |- | ||
| + | | | ||
| + | ::Add to them [the product of] the root of the triangle of the bottom base by the root of the triangle of the upper base, which is approximately 14 cubits minus a seventh; the total sum is 48 and a half. | ||
| + | |style="text-align:right;"|הוסף עליהם גדר משלש התושבת בגדר משלש הראש ויהיו י"ד אמה פחות שביעית על דרך קירוב יהיה הכל מ"ח וחצי | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(28-\frac{2}{7}\right)+\left[7-\left(\frac{1}{2}\sdot\frac{1}{7}\right)\right]+\left[\sqrt{28-\frac{2}{7}}\sdot\sqrt{7-\left(\frac{1}{2}\sdot\frac{1}{7}\right)}\right]=\left(28-\frac{2}{7}\right)+\left[7-\left(\frac{1}{2}\sdot\frac{1}{7}\right)\right]+\left(14-\frac{1}{7}\right)=48+\frac{1}{2}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | ::Multiply it by a third of the altitude, which is 2; the total is 97 and this is the volume of the truncated pyramid. | ||
| + | |style="text-align:right;"|מונה אותו בשליש הגובה והוא ב' יהיה הכל צ"ז והוא מדידת חתוכת הראש | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | ::<math>\scriptstyle{\color{blue}{\left[\left(28-\frac{2}{7}\right)+\left[7-\left(\frac{1}{2}\sdot\frac{1}{7}\right)\right]+\left[\sqrt{28-\frac{2}{7}}\sdot\sqrt{7-\left(\frac{1}{2}\sdot\frac{1}{7}\right)}\right]\right]\sdot\left(\frac{1}{3}\sdot6\right)=\left(48+\frac{1}{2}\right)\sdot2=97}}</math> | ||
| + | |- | ||
| + | |This way you know the volume of every truncated pyramid, whose bottom base is a pentagon, or other shape: | ||
| + | |style="text-align:right;"|ועל הדרך הזה תדע מדידת כל תמונה חתוכת הראש אשר תושבתה מחומש או תמונה אחרת | ||
| + | |- | ||
| + | |You know the areas of the two bases, add to them the product of the root of the area of the upper base by the root of the area of the bottom base, sum up all, then multiply [the sum] by a third of the altitude, and you find the volume of that shape. | ||
| + | |style="text-align:right;"|והוא שתהיה יודע השני ראשים בתשברתם ותוסיף עליהם רבוע גדר תשבורת הראש בגדר תשבורת התושבת ותכלול הכל ותכפול אותו בשליש הגובה ותמצא מדידת התמונה ההיא | ||
| + | |- | ||
| + | |I give you here examples of many shapes, so that you may train yourself in measuring them: | ||
| + | |style="text-align:right;"|והנה אתן לך דמיון בתמונות הרבה כדי שתרגיל את עצמך במדידתם | ||
| + | |- | ||
| + | !<span style=color:Green>Pyramid with a square base</span> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | *A pyramid, whose base is a square is measured as follows: each of the sides of the base is 24 cubits, and the lateral sides of the pyramid are 18 cubits. | ||
| + | |style="text-align:right;"|תמונה מחודדת תושבתה מרובע נמדדה כך יהיה כל אחד מצלעות התושבת מכ"ד אמות וצלעות המחודד מי"ח אמות | ||
| + | |- | ||
| + | | | ||
| + | :Take the square of 24; the result is 576. Take its half; it is 288. | ||
| + | |style="text-align:right;"|תקח מרובע הכ"ד וההוה תקע"ו קח מחציתם והם רפ"ח | ||
| + | |- | ||
| + | | | ||
| + | :Take also the square of 18; the result is 324. | ||
| + | |style="text-align:right;"|גם קח מרובע הי"ח וההוה שכ"ד | ||
| + | |- | ||
| + | | | ||
| + | :Subtract the 288 from it; 36 remains. Extract its root; it is 6 and this is the size of the altitude of the pyramid. | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{18^2-\left(\frac{1}{2}\sdot24^2\right)}=\sqrt{324-\left(\frac{1}{2}\sdot576\right)}=\sqrt{324-288}=\sqrt{36}=6}}</math> | ||
| + | |style="text-align:right;"|תגרע מאלה הרפ"ח נשארו ל"ו קח גדרם והם ו' וזהו שעור עמוד המחודד | ||
| + | |- | ||
| + | | | ||
| + | :Since the altitude is 6, we take its third; it is 2. Multiply it by 576; the result is 1152 and this is the volume of the pyramid. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(\frac{1}{3}\sdot6\right)\sdot576=2\sdot576=1152}}</math> | ||
| + | |style="text-align:right;"|ולפי שהעמוד הוא ו' נקח שלישיתו הם ב' ותכהו עם התקע"ו וההוה אלף קנ"ב וזהו תשבורת המחודד | ||
| + | |- | ||
| + | | | ||
| + | *A pyramid, whose base is a square, each of the sides of its base is 12 cubits, and its lateral sides are 36 cubits. | ||
| + | |style="text-align:right;"|<big>מחודד</big> שתושבתו מרובע אשר כל אחת מצלעות תושבתו בעל י"ב אמות וצלעות גבהותו מל"ו אמות | ||
| + | |- | ||
| + | | | ||
| + | :*We wish to find its altitude | ||
| + | |style="text-align:right;"|ונרצה למצוא העמוד | ||
| + | |- | ||
| + | | | ||
| + | ::We do as follows: | ||
| + | |style="text-align:right;"|נעשה כך | ||
| + | |- | ||
| + | | | ||
| + | ::We multiply the side of the base, i.e. the 12, by itself; the result is 144. | ||
| + | |style="text-align:right;"|נכפול צלע התושבת כלומ' הי"ב על עצמם וההוה קמ"ד | ||
| + | |- | ||
| + | | | ||
| + | ::We multiply also the other side by itself; the result is 144. | ||
| + | |style="text-align:right;"|עוד נכפול הצלע האחר על עצמו וההוה קמ"ד | ||
| + | |- | ||
| + | | | ||
| + | ::We sum them up; the result is 288. | ||
| + | |style="text-align:right;"|נחברם ביחד ההוה רפ"ח | ||
| + | |- | ||
| + | | | ||
| + | ::We extract its root; it is 16 and a little excess and this is the diagonal of the base. | ||
| + | ::<math>\scriptstyle{\color{blue}{\sqrt{12^2+12^2}=\sqrt{144+144}=\sqrt{288}=16+}}</math> | ||
| + | |style="text-align:right;"|נקח גדרם והם י"ו גם תוספת מעט וכך הוא קטר התושבת | ||
| + | |- | ||
| + | | | ||
| + | ::We take a half of 16; it is 8 and a half. | ||
| + | |style="text-align:right;"|נקח מחצית הי"ו והם ח' וחצי | ||
| + | |- | ||
| + | | | ||
| + | ::We multiply it by itself; the result is 72 and a quarter. | ||
| + | |style="text-align:right;"|ונכפלם על עצמם ההוה ע"ב ורביע | ||
| + | |- | ||
| + | | | ||
| + | ::We multiply also the 36, which is the lateral side, by itself; the result is 1296. | ||
| + | |style="text-align:right;"|עוד נכפול הל"ו שהם צלע גבהותו על עצמם ההוה אלף רצ"ו | ||
| + | |- | ||
| + | | | ||
| + | ::We subtract the 72 and a quarter from it; 1224 remains with a little excess. | ||
| + | |style="text-align:right;"|נגרע מאלה הע"ב ורביע וישארו אלף רכ"ד עם תוספת מעט שבם | ||
| + | |- | ||
| + | | | ||
| + | ::We extract its root; the result is 35 with a little excess and this is the length of the altitude. | ||
| + | |style="text-align:right;"|ונקח גדרם וההוה ל"ה עם תוספת מעט שבו וכן הוא אורך העמוד | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | ::<math>\scriptstyle{\color{blue}{\sqrt{36^2-\left[\frac{1}{2}\sdot\left(16+\right)\right]^2}=\sqrt{36^2-\left(8+\frac{1}{2}\right)^2}=\sqrt{1296-\left(72+\frac{1}{4}\right)}=\sqrt{1224+}=35+}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :Multiply it by 144, which is the area of the base; the result is 5040. | ||
| + | |style="text-align:right;"|תכה אלה עם הקמ"ד שהם תשבורת התושבת ההוה ה' אלפים ומ‫' | ||
| + | |- | ||
| + | | | ||
| + | :We take its third; the result is 1680 and this is the volume of the solid. | ||
| + | |style="text-align:right;"|נקח שלישית אלה ההוה אלף תר"פ וכן הוא תשבורת המוגשם | ||
| + | |- | ||
| + | |The reason we take the third is that every triangular prism is divided into three pyramids, whose altitudes are equal and their bases are triangles [Elements XII.3]. | ||
| + | |style="text-align:right;"|ולמה אנו לוקחים השלישית כי כל [מוגדר]‫<ref>P1031 om.</ref> מוגשם נחלק לג' מחודדים כשהם שוה הגובה ותושבותיהם ‫<ref>61v</ref>משולשים | ||
| + | |- | ||
| + | |However, we based our calculation on the [quadrangular] prism and the [quadrangular] prism is divided into two triangular prisms. So, every triangular prism with the altitude of the pyramid is three times half the given pyramid that have a square base. | ||
| + | |style="text-align:right;"|ואנו עשינו חשבוננו על מוגשם נכחי השטחים והמוגשם הנכחי נחלק לב' מגודרים וכל מגודר של עמוד של המחודד הוא ג' כפלי מחצית המחודד המונח והוא בעל תושבת מרובעת | ||
| + | |- | ||
| + | |As Euclid has demonstrated in Book 12 [Elements XII.7]. | ||
| + | |style="text-align:right;"|כן הראה זה במופת אקלידס במאמר [הי"ב]‫<ref>P1031 השני</ref> | ||
| + | |- | ||
| + | !<span style=color:Green>Truncated pyramid with a square base</span> | ||
| + | | | ||
| + | |- | ||
| + | |A truncated square pyramid, i.e. whose top is truncated, the sides of its bottom base are ten cubits, the sides of its upper base are 2 cubits, and its lateral sides are 9 cubits; we measure it as follows: | ||
| + | |style="text-align:right;"|<big>מחודד</big> מזונב כלומר חתוך הראש מרבע אשר צלעות תושבתו מעשר אמות וצלעות הראש מב' אמות וצלעות גבהותו מט' אמות נמדוד אותו כן | ||
| + | |- | ||
| + | | | ||
| + | ::Subtract the two cubits of the sides of the upper base from the sides of the bottom base; the remainder is 8. | ||
| + | |style="text-align:right;"|תגרע השני אמות של צלעות הראש מצלעות של התושבת וישארו ח‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Multiply it by itself; it is 64. | ||
| + | |style="text-align:right;"|תכפלם על עצמם ויהיו ס"ד | ||
| + | |- | ||
| + | | | ||
| + | ::We take its half; it is 32. | ||
| + | |style="text-align:right;"|נקח מחציתם והם ל"ב | ||
| + | |- | ||
| + | | | ||
| + | ::We multiply also the 9 by itself; it is 81. | ||
| + | |style="text-align:right;"|גם נכפול הט' על עצמם ויהיו פ"א | ||
| + | |- | ||
| + | | | ||
| + | ::We subtract 32 from it; the remainder is 49. | ||
| + | |style="text-align:right;"|נגרעם מאלה הל"ב ישארו מ"ט | ||
| + | |- | ||
| + | | | ||
| + | ::We extract its root; it is 7 and this is the altitude. | ||
| + | |style="text-align:right;"|נקח גדרם והם ז' וכן הוא העמוד | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | ::<math>\scriptstyle{\color{blue}{\sqrt{9^2-\frac{1}{2}\sdot\left(10-2\right)^2}=\sqrt{9^2-\left(\frac{1}{2}\sdot8^2\right)}=\sqrt{9^2-\left(\frac{1}{2}\sdot64\right)}=\sqrt{81-32}=\sqrt{49}=7}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :Since the altitude is 7 cubits, we find the volume of the solid as follows: | ||
| + | |style="text-align:right;"|ולפי שהעמוד ז' אמות נמצא תשבורת המוגשם כן | ||
| + | |- | ||
| + | | | ||
| + | ::We add the 2 of the upper base to the 10 of the bottom base; it is 12. | ||
| + | |style="text-align:right;"|נחבר הב' של הראש עם הי' של התושבת ויהיו י"ב | ||
| + | |- | ||
| + | | | ||
| + | ::We take its half; it is 6. | ||
| + | |style="text-align:right;"|ונקח חציים והם ו‫' | ||
| + | |- | ||
| + | | | ||
| + | ::We multiply it by itself; the result is 36. | ||
| + | |style="text-align:right;"|נכפלם על עצמם ויהיו ל"ו | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{\left[\frac{1}{2}\sdot\left(2+10\right)\right]^2=\left(\frac{1}{2}\sdot12\right)^2=6^2=36}}</math> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | ::Then, subtract 2 of the upper base from 10; the remainder is 8. | ||
| + | |style="text-align:right;"|אחר כך תגרע מהי' הב' של הראש וישארו ח‫' | ||
| + | |- | ||
| + | | | ||
| + | ::We take its half; it is 4. | ||
| + | |style="text-align:right;"|ונקח חציים והם ד‫' | ||
| + | |- | ||
| + | | | ||
| + | ::We multiply it by itself; the result is 16. | ||
| + | |style="text-align:right;"|ונכפלם על עצמם ויהיו י"ו | ||
| + | |- | ||
| + | | | ||
| + | ::We take its third; it is 5 and one third. | ||
| + | |style="text-align:right;"|ונקח שלישיתם יהיו ה' ושליש | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{1}{3}\sdot\left[\frac{1}{2}\sdot\left(10-2\right)\right]^2=\frac{1}{3}\sdot\left(\frac{1}{2}\sdot8\right)^2=\left(\frac{1}{3}\sdot16\right)=5+\frac{1}{3}}}</math> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | ::Add it to the 36; it is 41 and a third. | ||
| + | |style="text-align:right;"|הוסף אלה על הל"ו ויהיו מ"א ושליש | ||
| + | |- | ||
| + | | | ||
| + | ::Multiply it by 7; the result is 289 and one third; and this is the volume. | ||
| + | |style="text-align:right;"|כפלם על הז' ויהיו רפ"ט ושליש וכן הוא התשבורת | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | ::<math>\scriptstyle{\color{blue}{\left[\left[\frac{1}{2}\sdot\left(2+10\right)\right]^2+\frac{1}{3}\sdot\left[\frac{1}{2}\sdot\left(10-2\right)\right]^2\right]\sdot7=\left[36+\left(5+\frac{1}{3}\right)\right]\sdot7=\left(41+\frac{1}{3}\right)\sdot7=289+\frac{1}{3}}}</math> | ||
| + | |- | ||
| + | !<span style=color:Green>Pyramid with a right-angled triangular base</span> | ||
| + | | | ||
| + | |- | ||
| + | |If you wish to measure a pyramid whose base is a right-angled triangle, it is not necessary to investigate the lateral sides in order to find the altitude, because it has a right angle. | ||
| + | |style="text-align:right;"|<big>אם</big> תרצה למדוד מחודד אשר תושבתו משולש נצב אינו הכרח לחקור על צלעות הגבהות למצוא העמוד בהיותו בעל זוית נצבת | ||
| + | |- | ||
| + | | | ||
| + | *Let the altitude be 25 cubits, if one side of the right angle of the triangle's base is 4 cubits and the other is 5 cubits, do this: | ||
| + | |style="text-align:right;"|ויהיה אם העמוד כ"ה אמות ואם הראשונה שאצל הזוית שאצל תושבת המשולש ד' אמות ואם האחרת ה' אמות תעשה כך | ||
| + | |- | ||
| + | | | ||
| + | ::Multiply 4 by 5; the result is 20. | ||
| + | |style="text-align:right;"|כפול הד' על הה' ההוה כ‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Take its half; it is 10. | ||
| + | |style="text-align:right;"|קח חציים והם י‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Multiply this by the 25, which is the altitude; the result is 250. | ||
| + | |style="text-align:right;"|כפלם על כ"ה שהוא העמוד ההוה ר"נ | ||
| + | |- | ||
| + | | | ||
| + | ::Take its third; it is 41 and two thirds. | ||
| + | |style="text-align:right;"|קח שישיתם והם מ"א וב' שלישים | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{1}{6}\sdot\left[\left[\frac{1}{2}\sdot\left(4\sdot5\right)\right]\sdot25\right]=\frac{1}{6}\sdot\left[\left(\frac{1}{2}\sdot20\right)\sdot25\right]=\frac{1}{6}\sdot\left(10\sdot25\right)=\frac{1}{6}\sdot250=41+\frac{2}{3}}}</math> | ||
| + | |- | ||
| + | |Due to the reason that I mention, which is that every prism whose base is a triangle, which is half a square, is divided into three pyramids that have triangular bases, so is this prism. | ||
| + | |style="text-align:right;"|בעבור הסבה שאזכור והיא כל מגודר אשר תושבתו משלש בחצי מרבע הוא נחלק לג' מחודדים בעלי תושבות משולשים וכן המגודר הזה | ||
| + | |- | ||
| + | | | ||
| + | :As Euclid has shown in Book XII. | ||
| + | |style="text-align:right;"|כן הראה במופת אקלידס במאמר השני | ||
| + | |- | ||
| + | |The triangular prism is a half of a square [prism] and is divided into three pyramids, the result is six, [which is] a half and a third. | ||
| + | |style="text-align:right;"|והמגודר הוא חצי מרובע ונחלק לג' מחודדים ההוה הוא ו' וחצי ושליש | ||
| + | |- | ||
| + | |The pyramid with a triangular base is necessarily a sixth of the square [prism], so we take one sixth. | ||
| + | |style="text-align:right;"|ובהכרח שהמחודד בעל תושבת משלשי שלה ששית תושבת מהמרובע ונקח הששית | ||
| + | |- | ||
| + | !<span style=color:Green>Pyramid with an isosceles triangular base</span> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | *If the triangle is isosceles, the legs are 12 cubits, the base is 8 cubits, the lateral sides are 25 cubits, and you wish to measure the pyramid, do as follows: | ||
| + | |style="text-align:right;"|ואם המשולש שוה השוקים ויהיו השוקים מי"ב אמות והתושבת ח' אמות וצלעות הגבהות מכ"ה אמות ותרצה למדוד המחודד תעשה כך | ||
| + | |- | ||
| + | | | ||
| + | ::Divide the base, which is 8 cubits, into halves; the result is 4. | ||
| + | |style="text-align:right;"|תחלק התושבת שהוא ח' אמות לחצאין ההוה ד‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Multiply it by itself; the result is 16. | ||
| + | |style="text-align:right;"|כפלם על עצמם ההוה י"ו | ||
| + | |- | ||
| + | | | ||
| + | ::Multiply one of the sides by itself, that is 12 by itself; the result is 144. | ||
| + | |style="text-align:right;"|וכפול אחת הצלעות על עצמו כלומר הי"ב על עצמם ההוה קמ"ד | ||
| + | |- | ||
| + | | | ||
| + | ::Subtract the square of 4 from it, which is 16; the remainder is 128. | ||
| + | |style="text-align:right;"|גרע מאלה מרובע ד' שהם י"ו ישארו קכ"ח | ||
| + | |- | ||
| + | | | ||
| + | ::Extract the root, which is 11, a quarter, one part of 22, and one part of 44. This is the size of the height on the base of the isosceles triangle. | ||
| + | |style="text-align:right;"|קח גדרם שהם י"א ורביע וחלק מכ"ב וחלק ממ"ד וזהו השעור של העמוד אשר על תושבת המשלש שוה השוקים | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | ::<math>\scriptstyle{\color{blue}{\sqrt{12^2-\left(\frac{1}{2}\sdot8\right)^2}=\sqrt{12^2-4^2}=\sqrt{144-16}=\sqrt{128}=11+\frac{1}{4}+\frac{1}{22}+\frac{1}{44}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | ::If you wish to find the area, do as follows: multiply the altitude by the base, i.e. 11, a quarter, one part of 22, and one part of 44 by 8; the result is 90 and one part of 22. | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(11+\frac{1}{4}+\frac{1}{22}+\frac{1}{44}\right)\sdot8=90+\frac{1}{22}}}</math> | ||
| + | |style="text-align:right;"|ואם תרצה למצא התשברת תעשה כך תכפול העמוד על התושבת כלו' י"א ורביע וחלק מכ"ב וחלק ממ"ד על הח' ההוה צ' וחלק מכ"ב | ||
| + | |- | ||
| + | | | ||
| + | ::Multiply it by the altitude of the pyramid. | ||
| + | |style="text-align:right;"|כפלם על עמוד המחודד | ||
| + | |- | ||
| + | | | ||
| + | ::You find it as follows: since for every triangle we take a half of the altitude to the base, we take a half of the 11, one quarter, one part of 22, and one part of 44; the result is 5, a half, an eighth, one part of 44, and one part of 88. | ||
| + | |style="text-align:right;"|ותמצאנו כך לפי שבכל ‫<ref>62r</ref>משולש אנו לוקחים חצי עמוד התושבת נקח חצי הי"א ורביע וחלק מכ"ב וחלק ממ"ד והם ה' וחצי ושמינית וחלק ממ"ד וחלק מפ"ח | ||
| + | |- | ||
| + | | | ||
| + | ::We Multiply this by itself; the result is 32 and one part of 44. | ||
| + | |style="text-align:right;"|נכפלם על עצמם וההוה ל"ב וחלק ממ"ד | ||
| + | |- | ||
| + | | | ||
| + | ::We multiply also the lateral side, which is 25, by itself; the result is 625. | ||
| + | |style="text-align:right;"|גם נכפול צלעות הגבהות על עצמם שהם כ"ה ההווה תרכ"ה | ||
| + | |- | ||
| + | | | ||
| + | ::Subtract the 33 and [one part of 44]; the remainder is 593. | ||
| + | |style="text-align:right;"|תגרע הל"ב ורביע ההוה תקצ"ג | ||
| + | |- | ||
| + | | | ||
| + | ::We extract its root; the result is 24, a quarter and an eighth with a remainder and this is the size of the altitude. | ||
| + | |style="text-align:right;"|נקח גדרם והם כ"ד ורביע ושמיני' עם התוספת מעט וזהו שעור העמוד | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | ::<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle\sqrt{25^2-\left[\frac{1}{2}\sdot\left(11+\frac{1}{4}+\frac{1}{22}+\frac{1}{44}\right)\right]^2}&\scriptstyle=\sqrt{25^2-\left(5+\frac{1}{2}+\frac{1}{8}+\frac{1}{44}+\frac{1}{88}\right)^2}=\sqrt{625-\left(32+\frac{1}{44}\right)}\\&\scriptstyle=\sqrt{593}=24+\frac{1}{4}+\frac{1}{8}+\end{align}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | ::Multiply it by the area of the triangle, i.e. the 90 and one part of 22; the result is 2199. | ||
| + | |style="text-align:right;"|כפול אלה על תשבורת המשולש כלו' הצ' וחלק מכ"ב ההוה אלפים וקצ"ט | ||
| + | |- | ||
| + | | | ||
| + | ::We take from this a sixth of the square; the result is 367 and a half; and this is the volume of the pyramid. | ||
| + | |style="text-align:right;"|נקח מאלה ששית המרובע וההוה שס"ו וחצי וזהו תשבורת המחודד | ||
| + | |- | ||
| + | !<span style=color:Green>Pyramid with an obtuse-angled or acute angled triangular base</span> | ||
| + | | | ||
| + | |- | ||
| + | |If the pyramid is obtuse-angled and its base is an obtuse-angled triangle: multiply the area of the obtuse-angled triangle by the altitude, we take its third and you are left with the volume of the pyramid. | ||
| + | |style="text-align:right;"|ואם המחודד יהיה נרוח הזויות ויהיה תושבת משולש נרוח הזויות תכפול תשבורת המשלש נרוח הזויות עם העמוד ונקח השליש וישאר לך תשבורת המחודד | ||
| + | |- | ||
| + | |Likewise if it is acute-angled. | ||
| + | |style="text-align:right;"|וכן אם יהיה חד הזויות | ||
| + | |- | ||
| + | !<span style=color:Green>Pyramid with a right-angled triangular base</span> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | *A pyramid, whose base is a right-angled triangle, the height is 6 cubits, its base is 8 cubits, the hypotenuse that is opposite to the right angle is 10 cubits, and each of the lateral sides of the pyramid is 13 cubits. We wish to find its altitude. | ||
| + | |style="text-align:right;"|<big>מחודד</big> שתושבתו משלש נצב הזויות ויהיה עמוד ו' אמות ותושבתו ח' אמות והמיתר לזוית הנצבת י' אמות והמחודד כל אחת מצלעותיו י"ג אמות ונרצה למצוא עמודו | ||
| + | |- | ||
| + | | | ||
| + | :We do as follows: | ||
| + | |style="text-align:right;"|נעשה כך | ||
| + | |- | ||
| + | | | ||
| + | :First, we find the diameter of the circle that circumscribes the triangle, which is 10 cubits, and it is the hypotenuse that is opposite to the right angle. | ||
| + | |style="text-align:right;"|נמצא בתחלה אלכסון העגולה המקפת המשולש שיהיה י' אמות שהוא המיתר לזוית הנצבת | ||
| + | |- | ||
| + | | | ||
| + | :I take its half, which is 5, multiply it by itself; it is 25. | ||
| + | |style="text-align:right;"|ואקח חצייה והם ה' ואכפלם על עצמם וההוה כ"ה | ||
| + | |- | ||
| + | | | ||
| + | :I multiply the 13 of the lateral side by itself; the result is 169. | ||
| + | |style="text-align:right;"|גם אכפול הי"ג של צלע הגבהו' על עצמם וההוה קס"ט | ||
| + | |- | ||
| + | | | ||
| + | :I subtract the 25 from it; 144 remains. | ||
| + | |style="text-align:right;"|ואגרע מאלה הכ"ה וישארו קמ"ד | ||
| + | |- | ||
| + | | | ||
| + | :I extract its root; it is 12. The size [of the altitude] is found. | ||
| + | ::<math>\scriptstyle{\color{blue}{\sqrt{13^2-\left(\frac{1}{2}\sdot10\right)^2}=\sqrt{13^2-5^2}=\sqrt{169-25}=\sqrt{144}=12}}</math> | ||
| + | |style="text-align:right;"|ואקח גדרם והם י"ב והתשבורת נמצאה | ||
| + | |- | ||
| + | | | ||
| + | :Also, I first find the area of the triangle; it is 24 cubits. | ||
| + | |style="text-align:right;"|וכן בתחלה אמצא תשבורת המשולש והוא כ"ד אמות | ||
| + | |- | ||
| + | | | ||
| + | :I take a third of the altitude, which is the altitude of the pyramid; it is 4. | ||
| + | |style="text-align:right;"|ואקח שלישית העמוד שהוא עמוד המחודד והם ד‫' | ||
| + | |- | ||
| + | | | ||
| + | :Multiply it by the area of the base; it is 96 cubits and this is the volume of the pyramid. | ||
| + | ::<math>\scriptstyle{\color{blue}{24\sdot\left(\frac{1}{3}\sdot12\right)=24\sdot4=96}}</math> | ||
| + | |style="text-align:right;"|כפלם על תשבורת התושבת ויהיו צ"ו אמות וכן הוא תשבורת המחודד | ||
| + | |- | ||
| + | !<span style=color:Green>Pyramid with an equilateral triangular base</span> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | *A pyramid standing on an equilateral triangle - we measure it as follows: | ||
| + | |style="text-align:right;"|<big>מחודד</big> העומד על משולש שוה הצלעות נמדדהו כך | ||
| + | |- | ||
| + | | | ||
| + | :Let each of the sides of the base be 30 cubits and the lateral sides 20 cubits. | ||
| + | |style="text-align:right;"|יהיה כל צלע מצלעות התושבת מל' אמות וצלעות הגבהות כ' אמות | ||
| + | |- | ||
| + | | | ||
| + | :Multiply the 30 by itself; the result is 900. We take its third; it is 300. | ||
| + | |style="text-align:right;"|כפול הל' על עצמם וההוה תק"ת נקח שלישיתם והם ש‫' | ||
| + | |- | ||
| + | | | ||
| + | :We multiply also the [20] by itself; the result is 400. | ||
| + | |style="text-align:right;"|גם נכפול הת' על עצמם ההוה ת‫' | ||
| + | |- | ||
| + | | | ||
| + | :I subtract the 300 from it; 100 remains. Extract its root; it is 10 and this is the length of the altitude. | ||
| + | |style="text-align:right;"|ומאלה אגרע הש' נשארו ק' וקח גדרם והם י' וזהו אורך העמוד | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | ::<math>\scriptstyle{\color{blue}{\sqrt{20^2-\left(\frac{1}{3}\sdot30^2\right)}=\sqrt{400-\left(\frac{1}{3}\sdot900\right)}=\sqrt{400-300}=\sqrt{100}=10}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :Since the altitude is 10 cubits, the volume is found as follows: | ||
| + | |style="text-align:right;"|ולפי שהעמוד י' אמות ימצא התשבורת כן | ||
| + | |- | ||
| + | | | ||
| + | :We multiply the 30 by itself; the result is 900. We take its third and its tenth; it is 390. We take a third of it; it is 130. | ||
| + | |style="text-align:right;"|נכפול הל' על עצמם ההוה תת"ק נקח מאלה השלישית והעשירית והוא ש"צ נקח שלישית אלה והוא ק"ל | ||
| + | |- | ||
| + | | | ||
| + | :We multiply it by 10, which is the size of the altitude; the result is 1300 and this is the volume of the pyramid. | ||
| + | |style="text-align:right;"|נכפול אלה על י' שהוא שעור העמוד ההוה אלף וש' וזהו תשבורת המחודד | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | ::<math>\scriptstyle{\color{blue}{\left[\frac{1}{3}\sdot\left[\left(\frac{1}{3}+\frac{1}{10}\right)\sdot30^2\right]\right]\sdot10=\left[\frac{1}{3}\sdot\left[\left(\frac{1}{3}+\frac{1}{10}\right)\sdot900\right]\right]\sdot10=\left(\frac{1}{3}\sdot390\right)\sdot10=130\sdot10=1300}}</math> | ||
| + | |- | ||
| + | !<span style=color:Green>Pyramid with a pentagonal base</span> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | *A pyramid on pentagonal base, each of the sides of the base is 12 cubits and the lateral sides are 35 cubits. We want to find the altitude and the volume of the pyramid. | ||
| + | |style="text-align:right;"|<big>מחודד</big> על תושבת בעלת ה' זויות אשר כל אחת מצלעות התושבת מי"ב אמות וצלעות הגבהות מל"ה אמות ונרצה למצוא העמוד וגם תשבורת המחודד | ||
| + | |- | ||
| + | | | ||
| + | :I draw [a circle] around the pentagon, whose perimeter is 63 cubits. | ||
| + | |style="text-align:right;"|אחוק על הבעל ה' זויות אשר הקו המקיף ס"ג אמות | ||
| + | |- | ||
| + | | | ||
| + | :So, the diameter is 20 cubits. | ||
| + | |style="text-align:right;"|ואם כן האלכסון יהיה כ' אמות | ||
| + | |- | ||
| + | | | ||
| + | :Take half of it; it is 10. Multiply it by itself; the result is 100. | ||
| + | |style="text-align:right;"|קח מאלה חציים והם י' וכפלם על עצמם עלו ק‫' | ||
| + | |- | ||
| + | | | ||
| + | :Multiply also the 35 cubits of the lateral side by themselves; the result is 1225. | ||
| + | |style="text-align:right;"|עוד ‫<ref>62v</ref>כפול הל"ה אמות של גבהות הצלעות על עצמם יעלו אלף רכ"ה | ||
| + | |- | ||
| + | | | ||
| + | :Subtract from it the 100; 1125 remains. Extract its root; it is 33, a half, one part of 22, and a little excess, and this is the length of the altitude. | ||
| + | |style="text-align:right;"|גרע מאלה הק' ישארו אלף קכ"ה קח גדרם והם ל"ג וחצי וחלק מכ"ב עם תוספת וזהו אורך העמוד | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | ::<math>\scriptstyle{\color{blue}{\sqrt{35^2-\left(\frac{1}{2}\sdot20^2\right)}=\sqrt{35^2-10^2}=\sqrt{1225-100}=\sqrt{1125}=33+\frac{1}{2}+\frac{1}{22}+}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :Multiply it by the area of the pentagon. Do as follows: | ||
| + | |style="text-align:right;"|כפול תשבורת התושבת הבעלת ה' זויות וכן תעשה | ||
| + | |- | ||
| + | | | ||
| + | :Take half the 12 cubits of the [side of the base]; it is 6. Multiply it by itself; the result is 36. | ||
| + | |style="text-align:right;"|מן הי"ב אמות של התוספת קח חציים והם ו' וכפלם על עצמם וההוה ל"ו | ||
| + | |- | ||
| + | | | ||
| + | :We multiply also half the diameter, which is 10, by itself; the result is 100. | ||
| + | |style="text-align:right;"|עוד נכפול חצי האלכסון שהם י' על עצמם וההוה ק‫' | ||
| + | |- | ||
| + | | | ||
| + | :Subtract from it the 36; 64 remains. Extract its root; it is 8 and this is the size of the height of the triangle. | ||
| + | ::<math>\scriptstyle{\color{blue}{\sqrt{\left(\frac{1}{2}\sdot20^2\right)^2-\left(\frac{1}{2}\sdot12^2\right)}=\sqrt{10^2-6^2}=\sqrt{100-36}=\sqrt{64}=8}}</math> | ||
| + | |style="text-align:right;"|גרע מאלו הל"ו ונשארו ס"ד קח גדרם והם ח' והוא שעור העמוד של המשולש | ||
| + | |- | ||
| + | | | ||
| + | :Multiply it by [the side of] the base, which is 12; the result is 96. Take its half; it is 48 and this is the area of the triangle. | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{1}{2}\sdot\left(8\sdot12\right)=\frac{1}{2}\sdot96=48}}</math> | ||
| + | |style="text-align:right;"|כפלם עם התושבת שהם י"ב יעלה צ"ו קח חציים והם מ"ח והם תשבורת המשלש | ||
| + | |- | ||
| + | | | ||
| + | :Multiply it 5 times, since they are five triangles; it is 240 cubits. Multiply it by the altitude, which is 33, a half, and one part of 22; the result is 8050. | ||
| + | ::<math>\scriptstyle{\color{blue}{48\sdot\left(33+\frac{1}{2}+\frac{1}{22}\right)=8050}}</math> | ||
| + | |style="text-align:right;"|כפול אלה ה' פעמים אחרי שהם ה' משולשים ויהיו ר"מ אמות כפול אלה על העמוד שהוא ל"ג וחצי וחלק מכ"ב יעלו ח' אלפים ונ‫' | ||
| + | |- | ||
| + | | | ||
| + | :Take its sixth, which is [a sixth of the triangular prism]; the result is 1341 and 2-thirds; this is the volume of the pyramid. | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{1}{6}\sdot8050=1341+\frac{2}{3}}}</math> | ||
| + | |style="text-align:right;"|ומאלה קח ששיתם אחרי שהם ששה מגודרים ההוה אלף שמ"א וב' שלישים וזהו תשבורת המחודד | ||
| + | |- | ||
| + | |We can find the diagonal also without drawing the circle, since the side of the pentagon is a square of the side of the hexagon and the side of the decagon: | ||
| + | |style="text-align:right;"|ונוכל למצוא גם בלתי חקיקת העגולה את האלכסון לפי שצלע המחומש יש לו כח על צלע המשושה ועל צלע המעושר | ||
| + | |- | ||
| + | | | ||
| + | :We take half the side, i.e. a half of 12; it is 6. We multiply it by itself; the result is 36. | ||
| + | |style="text-align:right;"|נקח חצי הצלע כלומר חצי הי"ב ויהיו ו' נכפלם על עצמם יעלו ל"ו | ||
| + | |- | ||
| + | | | ||
| + | :We also multiply the 12 by itself; the result is 144. | ||
| + | |style="text-align:right;"|גם נכפול הי"ב על עצמם יעלו קמ"ד | ||
| + | |- | ||
| + | | | ||
| + | :Subtract the 36 from it; 108 remains. Extract its root; it is 10 cubits, a third and one part of 15 and this is the measure of the side of the hexagon and it is also the measure of the length from the center. | ||
| + | |style="text-align:right;"|גרע מאלה הל"ו נשארו ק"ח קח גדרם והוא י' אמות ושליש וחלק מט"ו וזהו שעור צלע בעל שש הזויות גם זהו שעור הארך מן המרכז | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | ::<math>\scriptstyle{\color{blue}{\sqrt{12^2-\left(\frac{1}{2}\sdot12\right)^2}=\sqrt{12^2-6^2}=\sqrt{144-36}=\sqrt{108}=10+\frac{1}{3}+\frac{1}{15}}}</math> | ||
| + | |- | ||
| + | !<span style=color:Green>Pyramid with a hexagonal base</span> | ||
| + | | | ||
| + | |- | ||
| + | |The measuring of a hexagonal [pyramid] is the same, without requiring the diagonal | ||
| + | |style="text-align:right;"|ויהיה גם מדידת בעל השש זויות כך בלתי שאדרוש את האלכסון | ||
| + | |- | ||
| + | | | ||
| + | *For example, let a hexagonal pyramid, each side of which is 12 cubits, the lateral sides are 35 cubits, and we wish to find the altitude and the volume of the pyramid, we do as follows: | ||
| + | |style="text-align:right;"|כגון שיהיה מחודד בעל שש זויות ויהיה כל א' מהצלעות מי"ב אמות וצלעות הגבהות מל"ה אמות ונרצה למצוא העמוד ותשבורת המחודד נעשה כך | ||
| + | |- | ||
| + | | | ||
| + | ::We multiply 12 by itself; the result is 144. | ||
| + | |style="text-align:right;"|נכפול הי"ב על עצמם יעלו קמ"ד | ||
| + | |- | ||
| + | | | ||
| + | ::35 by its self; the result is 1225 | ||
| + | |style="text-align:right;"|והל"ה על עצמם יעלו אלף רכ"ה | ||
| + | |- | ||
| + | | | ||
| + | ::Subtract 144 from it; the remainder is 1081 | ||
| + | |style="text-align:right;"|גרע מאלה הקמ"ד יעלו אלף פ"א | ||
| + | |- | ||
| + | | | ||
| + | ::We extract its root; it is 32 cubits, a half, a quarter, an eighth, and one part of 64; we know that this is the size of the altitude. | ||
| + | ::<math>\scriptstyle{\color{blue}{\sqrt{35^2-12^2}=\sqrt{1225-144}=\sqrt{1081}=32+\frac{1}{2}+\frac{1}{4}+\frac{1}{8}+\frac{1}{64}}}</math> | ||
| + | |style="text-align:right;"|נקח גדרם והוא ל"ב אמות וחצי ורביע ושמינית וחלק מס"ד וידענו שזהו שעור העמוד | ||
| + | |- | ||
| + | | | ||
| + | :Multiply it by the area of the hexagon. Extract it as follows: | ||
| + | |style="text-align:right;"|כפול אותו על תשבורת בעל השש וקח אותו כך | ||
| + | |- | ||
| + | | | ||
| + | :Since the hexagon consists of six equiangular triangles, take the area of one triangle and multiply it 6 times; you will find the area of the hexagon. | ||
| + | |style="text-align:right;"|לפי שבעל השש זויות יש לו ו' משולשים שוי הזויות קח תשבורת המשלש האחד וכפלהו ו' פעמים ותמצא תשבורת בעל הו' זויות | ||
| + | |- | ||
| + | | | ||
| + | :Do as follows with the equilateral triangle | ||
| + | |style="text-align:right;"|ועשה כן במשלש השוה הצלעות | ||
| + | |- | ||
| + | | | ||
| + | :Multiply its one side by itself; the result is 144. | ||
| + | |style="text-align:right;"|תכה צלעו הראשון על עצמו יעלה קמ"ד | ||
| + | |- | ||
| + | | | ||
| + | :We take its third; it is 48; and its tenth; it is 14, a third, and one part of 15. Sum them up; it is 62, a third, and one part of 15. | ||
| + | |style="text-align:right;"|נקח השליש והם מ"ח גם העשירית יהיו י"ד ושליש וחלק מט"ו חברם יהיו ס"ב ושליש וחלק מט"ו | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(\frac{1}{3}+\frac{1}{10}\right)\sdot12^2=\left(\frac{1}{3}\sdot144\right)+\left(\frac{1}{10}\sdot144\right)=48+\left(14+\frac{1}{3}+\frac{1}{15}\right)=62+\frac{1}{3}+\frac{1}{15}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :Multiply them 6 times, since they are six triangles; the result is 374, a quarter, and one part of 15. | ||
| + | ::<math>\scriptstyle{\color{blue}{6\sdot\left(62+\frac{1}{3}+\frac{1}{15}\right)=374+\frac{1}{4}+\frac{1}{15}}}</math> | ||
| + | |style="text-align:right;"|כפול אלה ו' פעמים אחרי שהם ו' משולשים יעלו שע"ד ורביע וחלק מט"ו | ||
| + | |- | ||
| + | | | ||
| + | :Multiply it by the altitude, which is 32, a half, a quarter, an eighth, and one part of 64; the result is 12414 and a third. | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(374+\frac{1}{4}+\frac{1}{15}\right)\sdot\left(32+\frac{1}{2}+\frac{1}{4}+\frac{1}{8}+\frac{1}{64}\right)=12414+\frac{1}{3}}}</math> | ||
| + | |style="text-align:right;"|כפול אלה על העמוד שהם ל"ב וחצי ורביע ושמינית וחלק מס"ד יעלו י"ב אלף תי"ד ושליש | ||
| + | |- | ||
| + | | | ||
| + | :Take its sixth, which is a sixth of the triangular prism; the result is 2052 and a third; this is the volume of the pyramid. | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{1}{6}\sdot\left(12414+\frac{1}{3}\right)=2052+\frac{1}{3}}}</math> | ||
| + | |style="text-align:right;"|קח ששיתם שהוא ששית המגודר יעלו ב' אלפים ונ"ב ושליש והם שעור המחודד | ||
| + | |- | ||
| + | !<span style=color:Green>Pyramid with an octagonal base</span> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | *A pyramid whose base is octagonal and we wish to measure it. | ||
| + | |style="text-align:right;"|<big>מחודד</big> אשר תושבתו בעל ח' זויות ונרצה למדוד אותה | ||
| + | |- | ||
| + | | | ||
| + | :Let it be a pyramid that each of the sides of its base is 10 cubits and its lateral sides are 15 cubits. | ||
| + | |style="text-align:right;"|יהיה מחודד אשר כל צלע מצלעות תושבתו מי' אמות וצלעות גבהותו מט"ו אמות | ||
| + | |- | ||
| + | | | ||
| + | :If you want to find the altitude and the volume of the pyramid, do as follows: | ||
| + | |style="text-align:right;"|ואם תרצה למצוא העמוד ותשבורת המחודד תעשה כך | ||
| + | |- | ||
| + | | | ||
| + | :Take half the side of the base of the octagon, i.e. half 10; it is 5. Multiply it by itself; it is 25. Double it; it is 50. Extract its root; it is 7 cubits and one part of 14. | ||
| + | |style="text-align:right;"|קח חצי צלע התושבת של בעל ח' זויות כלו' מהי' חציים ‫<ref>63r</ref>וההוה ה' וכפלם על עצמם וההוה כ"ה כפלם בשנים וההוה נ' קח גדרם והוא ז' אמות וחלק מי"ד | ||
| + | |- | ||
| + | | | ||
| + | :Add half the side of the octagon to them, which is 5; the sum is 12 and one part of 14. Multiply it by itself; the result is 146 plus a small excess. | ||
| + | |style="text-align:right;"|הוסף על אלה חצי צלע בעל שמנה תושבות שהם ה' ויהיה הסך י"ב וחלק מי"ד כפלם על עצמם ההוה קמ"ו עם תוספת מעט | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | ::<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle\left[\sqrt{2\sdot\left(\frac{1}{2}\sdot10\right)^2}+\left(\frac{1}{2}\sdot10\right)\right]^2&\scriptstyle=\left(\sqrt{2\sdot5^2}+5\right)^2=\left(\sqrt{2\sdot25}+5\right)^2=\left(\sqrt{50}+5\right)^2\\&\scriptstyle=\left[\left(7+\frac{1}{14}\right)+5\right]^2=\left(12+\frac{1}{14}\right)^2=146+\end{align}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :Multiply half the side by itself; it is 25. Add it to 146; the result is 171. Subtract 117; 54 remains. Extract its root; it is seven and a third and this is the size of the altitude. | ||
| + | |style="text-align:right;"|וכפול חצי הצלע על עצמו ההוה כ"ה חברם עם הקמ"ו וההוה קע"א גרע קי"ז וישארו נ"ד קח גדרם והם שבע ושליש וכן הוא שעור העמוד | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | ::<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle\sqrt{\left(\sqrt{2\sdot\left(\frac{1}{2}\sdot10\right)^2}+\left(\frac{1}{2}\sdot10\right)\right)^2+\left(\frac{1}{2}\sdot10\right)^2-117}&\scriptstyle=\sqrt{146+5^2-117}=\sqrt{146+25-117}\\&\scriptstyle=\sqrt{171-117}=\sqrt{54}=7+\frac{1}{3}\end{align}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :You find the volume of the pyramid as follows: | ||
| + | |style="text-align:right;"|והתשבורת של המחודד תמצאהו ככה | ||
| + | |- | ||
| + | | | ||
| + | :Take the area of the octagonal base, multiply it by the altitude; a third of the result is 1182 and this is the number of cubits in the volume of the pyramid with an octagonal base. | ||
| + | |style="text-align:right;"|קח תשבורת תושבת בעל הח' זויות וכפלה עם העמוד ושלישית ההוה הוא אלף וקפ"א וזהו שעור אמות תשבורת מחודד בעל ח' זויות | ||
| + | |- | ||
| + | !<span style=color:Green>Triangular pyramid with concavities</span> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | *Triangular pyramid with concavities, whose base consists of arcs that are less than a semicircle from end to end, the chord of each arc of the base is 10 cubits; the heights [of the arcs on these chords] are 2; and the lateral sides are 20. | ||
| + | |style="text-align:right;"|<big>מחודד</big> משולש בעל [מגרעות]‫<ref>P1031 מגבעות</ref> על תושבת קשתות קטנות מחצי העגולה מן הקצה אל הקצה ומיתר הקשת שבתושבת כל אחת י' אמות והעמודים הנופלים רחב ב' אמות וצלעות הגבהות מכ' אמות | ||
| + | |- | ||
| + | | | ||
| + | :We do as follows: | ||
| + | |style="text-align:right;"|ונעשה כך | ||
| + | |- | ||
| + | | | ||
| + | :We take half one of the chords of the base; it is 5. We multiply it by itself; the result is 25. | ||
| + | |style="text-align:right;"|נקח חצי מיתר אחת שבתושבת ויהיו ה' נכפלם על עצמם יעלו כ"ה | ||
| + | |- | ||
| + | | | ||
| + | :We multiply the other chord by itself; the result is 100. | ||
| + | |style="text-align:right;"|והמיתר האחר שהוא י' נכפלהו על עצמו וההוה ק‫' | ||
| + | |- | ||
| + | | | ||
| + | :Subtract the 25 from it; 75 remains. Extract its root; it is 8, a half, an eighth, and one part of 16, and this is the measure from the top of the triangle to the base, which is the height. | ||
| + | |style="text-align:right;"|גרע מאלה הכ"ה וישארו ע"ה קח גדרם והם ח' וחצי ושמינית וחלק א' מי"ו וכך הוא השעור שמראש המשולש עד התושבת והוא העמוד | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | ::<math>\scriptstyle{\color{blue}{\sqrt{10^2-\left(\frac{1}{2}\sdot10\right)^2}=\sqrt{10^2-5^2}=\sqrt{100-25}=\sqrt{75}=8+\frac{1}{2}+\frac{1}{8}+\frac{1}{16}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :We take their half, which is 4, a quarter, one part of 16, and one part of 32. Multiply them by themselves; the result is 16, a half, a quarter, and a ninth with a little excess and this is the size of half the base. | ||
| + | |style="text-align:right;"|נקח חציים והם ד' ורביע וחלק מי"ו וחלק מל"ב כפלם על עצמם ההוה י"ו וחצי ורביעית ותשיעית עם תוספת מעט וזהו שעור חצי התושבת | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | ::<math>\scriptstyle{\color{blue}{\left[\frac{1}{2}\sdot\left(8+\frac{1}{2}+\frac{1}{8}+\frac{1}{16}\right)\right]^2=\left(4+\frac{1}{4}+\frac{1}{16}+\frac{1}{32}\right)^2=16+\frac{1}{2}+\frac{1}{4}+\frac{1}{9}+}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :Multiply 5 by itself; the result is 25. The total sum is 43, a half, a quarter, and a ninth. Take their root; it is 6, a half, and a ninth, and this is [the distance] from the center of the circle that circumscribes the triangle. | ||
| + | |style="text-align:right;"|כפול הה' על עצמם ההוה כ"ה והסך ביחד יהיו מ"ג וחצי ורביעית ותשיעית קח גדרם והוא ו' וחצי ותשיעית וכן הוא ממרכז העגולה המקפת המשלש | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | ::<math>\scriptstyle{\color{blue}{\sqrt{\left(16+\frac{1}{2}+\frac{1}{4}+\frac{1}{9}+\right)+5^2}=\sqrt{\left(16+\frac{1}{2}+\frac{1}{4}+\frac{1}{9}+\right)+25}=\sqrt{43+\frac{1}{2}+\frac{1}{4}+\frac{1}{9}}=6+\frac{1}{2}+\frac{1}{9}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :When you want to find the altitude, do as follows: | ||
| + | |style="text-align:right;"|וכשתרצה למצוא העמוד תעשה כן | ||
| + | |- | ||
| + | | | ||
| + | :Multiply the 6, a half, and a ninth by themselves; the result is 43, a half, a quarter, and a ninth. | ||
| + | |style="text-align:right;"|כפול הו' וחצי ותשיעית על עצמם ההוה מ"ג אמות וחצי ורביעית ותשיעי‫' | ||
| + | |- | ||
| + | | | ||
| + | :Multiply also the lateral side, which is 20, by itself; the result is 400. | ||
| + | |style="text-align:right;"|גם כפול צלעות הגבהות שהם כ' על עצמם ההוה ת‫' | ||
| + | |- | ||
| + | | | ||
| + | :Subtract the 43, a half, a quarter, and a ninth from it; 356 and one part of 18 remain. take their root; it is 18, a half, a quarter, and a ninth, and this is the size of the altitude. | ||
| + | |style="text-align:right;"|גרע מאלה המ"ג וחצי ורביעית ותשיעית ישארו שנ"ו וחלק מי"ח קח גדרם והוא י"ח וחצי ורביעית ותשיעית וכן הוא שעור העמוד | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | ::<math>\scriptstyle{\color{blue}{\sqrt{20^2-\left(6+\frac{1}{2}+\frac{1}{9}\right)^2}=\sqrt{400-\left(43+\frac{1}{2}+\frac{1}{4}+\frac{1}{9}\right)}=\sqrt{356+\frac{1}{18}}=18+\frac{1}{2}+\frac{1}{4}+\frac{1}{9}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :Multiply it by the triangular base, and this is how you extract it: | ||
| + | |style="text-align:right;"|כפלהו עם תושבת המשולש וכך תקחהו | ||
| + | |- | ||
| + | | | ||
| + | :[Multiply] the 5, which is half [the chord of] the base by the height of the triangular base, which is 8, a half, an eighth, and one part of 16; the result is 43 and a half and this is the number of cubits that are the area of the triangle. | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(\frac{1}{2}\sdot10\right)\sdot\left(8+\frac{1}{2}+\frac{1}{8}+\frac{1}{16}\right))=5\sdot\left(8+\frac{1}{2}+\frac{1}{8}+\frac{1}{16}\right)=43+\frac{1}{2}}}</math> | ||
| + | |style="text-align:right;"|הה' שהם מחצית התושבת על עמוד תושבת המשולש שהם ח' וחצי ושמינית וחלק א' מי"ו ההוה מ"ג וחצי וכן הוא שעור אמות התשבורת המשולש | ||
| + | |- | ||
| + | | | ||
| + | :Multiply it by 1[8], a half, a quarter, and a ninth; the result is 820 plus a little excess and this is the volume of the triangular [prism]. | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(43+\frac{1}{2}\right)\sdot\left(18+\frac{1}{2}+\frac{1}{4}+\frac{1}{9}\right)=820+}}</math> | ||
| + | |style="text-align:right;"|כפלם על י"א וחצי ורביע ותשיעית ההוה תת"כ עם תוספת מעט וזהו תשבורת המשולש | ||
| + | |- | ||
| + | | | ||
| + | :From this the concavities should be subtracted. Do as follows: | ||
| + | |style="text-align:right;"|ומזה ראוי להוציא המגרעות וכן תעשה | ||
| + | |- | ||
| + | | | ||
| + | :Sum up [the chord of] the base and the height [of the arc on the chord], 10 and 2; it is 12 cubits. Multiply them by the altitude of the pyramid, which is 1[8], a half, a quarter, and a ninth; the result is 226 cubits. Multiply them by 3, since there are three concavities; the result is 679. | ||
| + | |style="text-align:right;"|תחבר התושבת והעמוד הי' והב' וההוא י"ב אמות כפלם עם עמוד המחודד שהם י"א וחצי ורביע ותשיעית ההוה רכ"ו אמות כפלם על ג' לפי ששם ג' מגרעות ההוה תרע"ט | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | ::<math>\scriptstyle{\color{blue}{3\sdot\left[\left(10+2\right)\sdot\left(18+\frac{1}{2}+\frac{1}{4}+\frac{1}{9}\right)\right]=3\sdot\left[12\sdot\left(18+\frac{1}{2}+\frac{1}{4}+\frac{1}{9}\right)\right]=3\sdot226=679}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :The whole complement is 820 cubits. We subtract the 679 from them; 141 remains. We take a sixth of it, because this is the triangular prism; it is 23 and [a half] and this is the volume of the shape. | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{1}{6}\sdot\left(820-679\right)=\frac{1}{6}\sdot141=23+\frac{1}{2}}}</math> | ||
| + | |style="text-align:right;"|וכל התשלום הוא תת"כ אמות נגרע מאלה התרע"ט ונשארו קמ"א נקח מאלה הששית ההוה כ"ג ושלישים ב' אחרי שהם מהמגודר הם כ"ג וב' שלישים וזהו תשבורת התמונה | ||
| + | |- | ||
| + | |It has already been explained in Book 12 of the Elements that every prism with a triangular base is divided into three equal pyramids. | ||
| + | |style="text-align:right;"|וכבר התבאר ב[[ספר_היסודות_לאקלידס#Elements_XII_6|מאמר הי"ב מספר היסודות]] שכל מגודר שתושבתו משולשת יחלק לג' מחודדים שוים | ||
| + | |- | ||
| + | |It is known that every pyramid is a third of a prism that has the same sides and the same altitude. | ||
| + | |style="text-align:right;"|‫<ref>63v</ref>וידוע שכל מחודד הוא שלישית המגודר כשיהיו בעלי צלעות שוות וגובה שוה | ||
| + | |- | ||
| + | |It is clear from this that any pyramid with any base is a third of the prism whose base is equal to its base and its altitude is equal to its altitude. | ||
| + | |style="text-align:right;"|ומבואר מזה שכל מחודד שיהיה על איזו תמונה שיהיה הוא שלישית המוגשם הנכחי שתושבתו שוה לתושבתו וגבהו הוא שוה לגבהו | ||
| + | |- | ||
| + | !<span style=color:Green>"Mishko"</span> | ||
| + | | | ||
| + | |- | ||
| + | |The shape called in Greek "mishko", whose upper base and bottom base are scalene, is measured as follows: | ||
| + | |style="text-align:right;"|<big>התמונה</big> הנקראת בלשון יון מישקו והיא תמונה שהיא מתחלפת הצלעות צלעות התושבת גם הראש נמדדה כך | ||
| + | |- | ||
| + | | | ||
| + | :The height is 50 cubits. | ||
| + | |style="text-align:right;"|הגובה יהיה נ' אמות | ||
| + | |- | ||
| + | | | ||
| + | :If the long side of the base of this shape is 24 cubits and the short [side] is 16 cubits. | ||
| + | |style="text-align:right;"|ואם תושבת התמונה צלעה האחת הגדולה בעלת כ"ד אמות והקטן בעל י"ו אמות | ||
| + | |- | ||
| + | | | ||
| + | :If the long side of the top is 12 cubits and the short [side] is 8 cubits. | ||
| + | |style="text-align:right;"|ואם הראש הצלע הגדולה בעלת י"ב אמות ואם הקטנה בעלת ח' אמות | ||
| + | |- | ||
| + | | | ||
| + | :We sum up the long sides of the top and the base, meaning the 12 and the 24; it is 36. | ||
| + | |style="text-align:right;"|נחבר הצלעו' הגדולו' של הראש ושל התושבת כלומר הי"ב הכ"ד ההוה ל"ו | ||
| + | |- | ||
| + | | | ||
| + | :I sum up also the short sides of the top and the base, meaning the 16 and the 8; it is 24. | ||
| + | |style="text-align:right;"|וכן חברתי הצלעות הקטנות של הראש ושל התושבת כלומר הי"ו והח' וההוה כ"ד | ||
| + | |- | ||
| + | | | ||
| + | :Take half the 36; it is 18; also half the 24; it is 12. Multiply it by 18; the result is 216. | ||
| + | ::<math>\scriptstyle{\color{blue}{\left[\frac{1}{2}\sdot\left(12+24\right)\right]\sdot\left[\frac{1}{2}\sdot\left(16+8\right)\right]=\left(\frac{1}{2}\sdot36\right)\sdot\left(\frac{1}{2}\sdot24\right)=\left(18\sdot12\right)=216}}</math> | ||
| + | |style="text-align:right;"|קח חצי הל"ו וההוה י"ח וכן חצי הכ"ד וההוה י"ב כפול אלה על י"ח וההוה רי"ו | ||
| + | |- | ||
| + | | | ||
| + | :We subtract the long side of 12 from the 24; 12 remains. We take its half; it is 6. | ||
| + | |style="text-align:right;"|ועוד נגרע מן הצלע הגדולה הי"ב מן הכ"ד וישארו י"ב נקח חציים והם ו‫' | ||
| + | |- | ||
| + | | | ||
| + | :We subtract the side of the top from [the side of] the base, meaning the short side, which is 8, from 16; 8 remains. We take its half; it is 4. | ||
| + | |style="text-align:right;"|נגרע צלע הראש מן התושבת כלומ' הצלע הקטן והם הח' מן הי"ו וישארו ח' נקח חציים ויהיו ד‫' | ||
| + | |- | ||
| + | | | ||
| + | :We multiply it by 6; the result is 24. Take its third; it is 8. | ||
| + | |style="text-align:right;"|כפלם על ו' ההוה כ"ד קח השלישית והם ח‫' | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{1}{3}\sdot\left[\left[\frac{1}{2}\sdot\left(24-12\right)\right]\sdot\left[\frac{1}{2}\sdot\left(16-8\right)\right]\right]=\frac{1}{3}\sdot\left[\left(\frac{1}{2}\sdot12\right)\sdot\left(\frac{1}{2}\sdot8\right)\right]=\frac{1}{3}\sdot\left(6\sdot4\right)=\left(\frac{1}{3}\sdot24\right)=8}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :We add it to 216; the sum is 224. | ||
| + | |style="text-align:right;"|ונוסיפם על הרי"ו ויהיו יחד רכ"ד | ||
| + | |- | ||
| + | | | ||
| + | :We multiply it by the height, which is 50; the result is 11200 and this is the volume of this shape. | ||
| + | |style="text-align:right;"|כפלם על הגובה שהם נ' ההוה י"א אלף ר' והם שעור תשבורת זאת התמונה | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | ::<math>\scriptstyle{\color{blue}{\begin{align}&\scriptstyle\left[\left[\left[\frac{1}{2}\sdot\left(12+24\right)\right]\sdot\left[\frac{1}{2}\sdot\left(16+8\right)\right]\right]+\frac{1}{3}\sdot\left[\left[\frac{1}{2}\sdot\left(24-12\right)\right]\sdot\left[\frac{1}{2}\sdot\left(16-8\right)\right]\right]\right]\sdot50\\&\scriptstyle=\left(216+8\right)\sdot50=224\sdot50=11200\\\end{align}}}</math> | ||
| + | |- | ||
| + | |We measure it this way even if it has different numbers. | ||
| + | |style="text-align:right;"|וכן נמדדהו גם אם היה בעל [מספרים]‫<ref>P1031: משפטים</ref> אחרים | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== Chapter Two on the Measuring of the Spheres and Round Solids ==== | ||
| + | |||
| + | |style="width:45%; text-align:right;"|<big>הפרק השני</big> במדידת הכדורים והגופים העגולים | ||
| + | |- | ||
| + | |The sphare: Euclid has defined it in Book Eleven of the Elements that "it is when the semicircle is drawn round with the diameter fixed in two points, so they do not move, and the arc, which is half the perimeter, revolves until it returns to its position; it is the circular solid. The center of the the sphare and the center of the circle are the same". | ||
| + | |style="text-align:right;"|[[ספר_היסודות_לאקלידס#Elements_XI_def_sphere|הכדור]] גדרו אקלידס במאמר אחד עשר מספר היסודות שהוא אשר יעבור חצי עגולה כאשר יקויים קו הקוטר בשני צירים עד שלא יסורו וסבבה הקשת אשר היא חצי הקו המקיף עד שתשוב אל מקומם והוא מוגשם העגול ומרכז הכדור ומרכז העגולה אחת | ||
| + | |- | ||
| + | !<span style=color:Green>Sphere</span> | ||
| + | | | ||
| + | |- | ||
| + | |One can measure the area of the surface surrounding the sphere alone, or measure the volume of the whole solid. | ||
| + | |style="text-align:right;"|והכדור יתכן למדוד התשבורת של השטח המקיף אותו לבד גם יתכן למדוד תשבורת כל גופו | ||
| + | |- | ||
| + | |When we want to find the area of the surface, square the diameter of the sphere, multiply this square by three and one-seventh, and you will get the area of the surface of the sphere. | ||
| + | |style="text-align:right;"|וכאשר נרצה למצוא תשבורת השטח תרבע את קטר הכדור ותכפול את המרובע הזה ג' פעמים ושביעית פעם ויעלה בידך משיחת שטח הכדור | ||
| + | |- | ||
| + | |Multiply if by a sixth of the diameter and you will receive the volume of the sphere. | ||
| + | |style="text-align:right;"|כפלהו בשתות הקטר ויעלה בידך תשבורת גוף הכדור | ||
| + | |- | ||
| + | | | ||
| + | *As a sphere whose diameter is 7 cubits, the perimeter is 22 cubits and we wish to find its volume. | ||
| + | |style="text-align:right;"|כגון ז' אמות כדור בקטרו והקו המקיף כ"ב אמות ונרצה למצוא תשבורת גופו | ||
| + | |- | ||
| + | | | ||
| + | :We do as follows: | ||
| + | |style="text-align:right;"|נעשה כך | ||
| + | |- | ||
| + | | | ||
| + | :We multiply the diameter by itself; the result is 49. When you multiply this square by 3 and one-seventh, it will be 154 and these are the cubits of the surface of the sphere. | ||
| + | :<math>\scriptstyle{\color{blue}{7^2\sdot\left(3+\frac{1}{7}\right)=49\sdot\left(3+\frac{1}{7}\right)=154}}</math> | ||
| + | |style="text-align:right;"|נכפול הקטר על עצמו ויעלו מ"ט וכשתכפול זה המרובע ג' פעמים ושביעית פעם יהיה קנ"ד והן אמות שטח הכדור | ||
| + | |- | ||
| + | | | ||
| + | :Multiply them by a sixth of the diameter, which is 1 and a sixth; it is 180 minus a third and this is the volume of this sphere. | ||
| + | :<math>\scriptstyle{\color{blue}{154\sdot\left(1+\frac{1}{6}\right)=180-\frac{1}{3}}}</math> | ||
| + | |style="text-align:right;"|כפול אותן בשתות הקטר והוא א' ושתות יהיה ק"פ פחות שלש והוא תשבורת הכדור הזה | ||
| + | |- | ||
| + | | | ||
| + | *Another way: a sphere whose diameter is 7 cubits, the perimeter is 22 cubits and we wish to find its volume. | ||
| + | |style="text-align:right;"|<big>דרך אחרת</big> כדור אשר קטרו ז' אמות והקו המקיף כ"ב ‫<ref>64r</ref>אמות ונרצה למצוא תשבורת גופו | ||
| + | |- | ||
| + | | | ||
| + | :We do as follows: | ||
| + | |style="text-align:right;"|נעשה כך | ||
| + | |- | ||
| + | | | ||
| + | :We multiply the diameter by itself; the result is 49. We multiply it again by the diameter, which is 7; the result is 343 cubits. We multiply them 11 times; they are 3773 cubits. We divide them by 21; the resulting quotient is 179 and 2-thirds and this is the volume of the sphere. | ||
| + | :<math>\scriptstyle{\color{blue}{\frac{7^2\sdot7\sdot11}{21}=\frac{49\sdot7\sdot11}{21}=\frac{343\sdot11}{21}=\frac{3773}{21}=179+\frac{2}{3}}}</math> | ||
| + | |style="text-align:right;"|נכפול הקטר על עצמו וההוה מ"ט עוד נכפול אלה על הקטר שהם ז' יעלו שמ"ג אמות נכפול אלה י"א פעם יהיו ג' אלפים תשע"ג אמות נחלקם על כ"א ויעלה החלק האחד קע"ט וב' שלישים וכן שעור תשבורת גוף הכדור | ||
| + | |- | ||
| + | | | ||
| + | :If you want to measure the surface, do as follows: | ||
| + | |style="text-align:right;"|ואם תרצה למדוד השטח תעשה כך | ||
| + | |- | ||
| + | | | ||
| + | :We always find the diameter, which is 7 in our example, and multiply it by the perimeter, which is 22; the result is 154 and this is the area of the surface of the sphere. | ||
| + | :<math>\scriptstyle{\color{blue}{7\sdot22=154}}</math> | ||
| + | |style="text-align:right;"|נמצא לעולם הקטר והם במשלינו ז' ונכפלם עם המקיף שהם כ"ב ההוה קנ"ד וכן הוא תשבורת שעור שטח הכדור | ||
| + | |- | ||
| + | |If you wish to calculate the air enclosed by the sphere, according to the explanation we have preceded concerning the measuring of the sphere without the thickness of the sphere walls. | ||
| + | |style="text-align:right;"|<big>אם</big> תרצה למדוד האויר המוקף בכדור תמדוד לפי הביאור אשר הקדמנו במדידת הכדור בלתי עובי הכתלים | ||
| + | |- | ||
| + | | | ||
| + | *As when the length of the diameter of the sphere's airspace is 8 cubits and the thickness of the walls is 2 cubits. | ||
| + | |style="text-align:right;"|כגון שיהיה אורך קטר האויר מהכדור ח' אמות ועובי שתי הכתלים ב' אמות | ||
| + | |- | ||
| + | | | ||
| + | :Multiply the 8 cubits of the diameter of the airspace by themselves; the result is 64. Multiply it again by the 8 of the diameter; the result is 512 cubits. Multiply them by 11; the result is 5632. Divide it by 21; the result is 268 cubits, one-seventh and one part of 21, and this is the volume of the airspace. | ||
| + | :<math>\scriptstyle{\color{blue}{\frac{8^2\sdot8\sdot11}{21}=\frac{64\sdot8\sdot11}{21}=\frac{512\sdot11}{21}=\frac{5632}{21}=268+\frac{1}{7}+\frac{1}{21}}}</math> | ||
| + | |style="text-align:right;"|כפול הח' אמות של קטר האויר על עצמם יעלו ס"ד כפול אלה עוד על הח' של הקטר יעלו תקי"ב אמו' כפלם על י"א יעלו ה' אלפים תרל"ב חלקם על כ"א יעלו רס"ח אמות ושביעית וחלק מן כ"א וזהו תשבורת אויר הכדור | ||
| + | |- | ||
| + | !<span style=color:Green>Hemisphere</span> | ||
| + | | | ||
| + | |- | ||
| + | |If you wish to measure a hemisphere, measure it according to the explanation of the measurement of the sphere, then divide the product by 42. | ||
| + | |style="text-align:right;"|<big>אם</big> תרצה למדוד חצי כדור תמדדהו לפי באור מדידת הכדור והנקבצים תחלקם על מ"ב | ||
| + | |- | ||
| + | | | ||
| + | *Example: the diameter is 7 cubits, the perimeter is 22 cubits and you wish to find the volume of the hemisphere. | ||
| + | |style="text-align:right;"|דמיון הקטר ז' אמות והקו המקיף כ"ב אמות ותרצה למצוא תשבורת גופני של חצי הכדור | ||
| + | |- | ||
| + | | | ||
| + | :Do as follows: | ||
| + | |style="text-align:right;"|תעשה כן | ||
| + | |- | ||
| + | | | ||
| + | :Multiply the 7 cubits of diameter by themselves; the result is 49. Multiply them again by the 7 cubits of diameter; the result is 343 cubits. Multiply them by 11, then divide the product by 42; the result is 89, a half and one-third and this is the volume of the hemisphere. | ||
| + | :<math>\scriptstyle{\color{blue}{\frac{7^2\sdot7\sdot11}{42}=\frac{49\sdot7\sdot11}{42}=\frac{343\sdot11}{42}=89+\frac{1}{2}+\frac{1}{3}}}</math> | ||
| + | |style="text-align:right;"|כפול הז' אמות של הקטר על עצמם ההוה מ"ט כפול עוד אלה על הז' אמות של הקטר ההוה שמ"ג כפול אלה על י"א וחלק העולה על מ"ב וההוה פ"ט אמות וחצי ושליש וכן הוא שעור חצי הכדור | ||
| + | |- | ||
| + | |If you want to measure [the volume of] the hemisphere's airspace, measure it according to the explanation preceded regarding the measurement of the hemisphere without the thickness of the walls. | ||
| + | |style="text-align:right;"|<big>אם</big> תרצה למדוד אויר חצי הכדור תמדדהו לפי הביאור אשר קדם במדידת תשבורת חצי הכדור בלתי עובי הכתלים | ||
| + | |- | ||
| + | | | ||
| + | *Example: the diameter of the hemisphere's airspace is 10 cubits and the thickness of the walls is 4 cubits. | ||
| + | |style="text-align:right;"|דמיון יהיה קטר אויר חצי הכדור י' אמות ועובי שתי הכתלים ד' אמות | ||
| + | |- | ||
| + | | | ||
| + | :Multiply the 10 cubits of the diameter of the airspace alone by themselves; the result is 100. Multiply the 100 again by the 10 mentioned; the result is 1000. Multiply it by 11; the result is 11000. Divide it by 42; the result is 261 cubits, a half, one-third and one part of 14, and this is the volume of the hemisphere's airspace. | ||
| + | :<math>\scriptstyle{\color{blue}{\frac{10^2\sdot10\sdot11}{42}=\frac{100\sdot10\sdot11}{42}=\frac{1000\sdot11}{42}=\frac{11000}{42}=261+\frac{1}{2}+\frac{1}{3}+\frac{1}{14}}}</math> | ||
| + | |style="text-align:right;"|כפל י' אמות קטר האויר לבד על עצמם וההוה ק' עוד כפול הק' על הי' הנזכרים ההוה אלף כפלם על י"א ההוה אלפים י"א חלקם על מ"ב ההוה רס"א אמות וחצי ושלישית חלק מי"ד וזהו שעור אמות אויר חצי הכדור | ||
| + | |- | ||
| + | !<span style=color:Green>Quarter of a sphere</span> | ||
| + | | | ||
| + | |- | ||
| + | |If you wish to measure a quarter of a sphere, measure it according to the explanation we have preceded of the measurement of the hemisphere, then divide the product by 84. | ||
| + | |style="text-align:right;"|<big>אם</big> תרצה למדוד רביע הכדור תמדוד לפי הבאור שהקדמנו במדידת חצי הכדור והנקבצים חלקם על פ"ד | ||
| + | |- | ||
| + | | | ||
| + | *Example: the diameter of the quarter of the sphere with the thickness of the walls is 14 cubits. | ||
| + | |style="text-align:right;"|דמיון קטר הרביע עם עובי ב' כתלים [י"ד]‫<ref>P1031 ד'</ref> אמות | ||
| + | |- | ||
| + | | | ||
| + | :Multiply them by themselves; the result is 196. Multiply them by the diameter mentioned; the result is 2744. Multiply them by 11; the result is 30184. Divide them by 84 and take the quotient; the result is 359 and one-third and this is the volume of the quarter of the sphere. | ||
| + | :<math>\scriptstyle{\color{blue}{\frac{14^2\sdot14\sdot11}{84}=\frac{196\sdot14\sdot11}{84}=\frac{2744\sdot11}{84}=\frac{30184}{84}=359+\frac{1}{3}}}</math> | ||
| + | |style="text-align:right;"|כפלם על עצמם ההוה קצ"ו כפול אלה על י"ד של הקטר הנזכר ההוה ב' אלפים תשמ"ד כפלם על י"א ההוה ל' אלף וקפ"ד חלקם על פ"ד וקח החלק האחד וההוה הוא שנ"ט ושלישית וכן הוא שעור רביע הכדור | ||
| + | |- | ||
| + | |If you want to measure the airspace of the mentioned quarter and find its volume, measure it according to the explanation mentioned regarding the measurement of the quarter without the thickness of the walls. | ||
| + | |style="text-align:right;"|<big>אם</big> תרצה למדוד אויר הרביע הנז' ולמצוא תשברתו הגופני תמדוד לפי הביאור הנזכר ‫<ref>64v</ref>במדידת הרביע בלתי עובי הכתלים | ||
| + | |- | ||
| + | | | ||
| + | :Meaning the 10 cubits of the diameter of the airspace; the result is 100. Multiply the 100 again by the 10; the result is one thousand. Multiply the thousand by 11; the result is 11000. Divide it by 84; the result is 130 cubits, a half, one-third, one part of 12 and one part of 28, and this is the volume of the quarter's airspace. | ||
| + | |style="text-align:right;"|כלומר הי' אמות של קטר האויר ויהיו ק' עוד כפול הק' על הי' ההוה אלף כפול האלף על י"א ההוה י"א אלפים חלקם על פ"ד ההוה ק"ל אמות וחצי ושליש וחלק מי"ב וחלק מכ"ח וכן הוא שעור מדידת אויר [הרביע]‫<ref>P1031 ורביע</ref> | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{\frac{10^2\sdot10\sdot11}{84}=\frac{100\sdot10\sdot11}{84}=\frac{1000\sdot11}{84}=\frac{11000}{84}=130+\frac{1}{2}+\frac{1}{3}+\frac{1}{12}+\frac{1}{28}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :Subtract them from 359 and one-third; 228 cubits remain, a quarter and an eighth, and this is the required volume [of the walls]. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(359+\frac{1}{3}\right)-\left(130+\frac{1}{2}+\frac{1}{3}+\frac{1}{12}+\frac{1}{28}\right)=228+\frac{1}{4}+\frac{1}{8}}}</math> | ||
| + | |style="text-align:right;"|גרעם מהשנ"ט ושליש הנחברים וישארו רכ"ח אמות ורביע ושמינית וזהו תשבורת המבוקש | ||
| + | |- | ||
| + | !<span style=color:Green>Hemispherical pool</span> | ||
| + | | | ||
| + | |- | ||
| + | |If you want to measure a round spherical pool that is hemispherical, I have already demonstrated to you its measurement by measuring the hemisphere. | ||
| + | |style="text-align:right;"|<big>אם</big> רצית למדוד בריכה מעוגלת כדוריית והיא חצי כדור כבר הראיתיך מדידתו במדידת חצי הכדור | ||
| + | |- | ||
| + | !<span style=color:Green>Pool smaller than a hemisphere</span> | ||
| + | | | ||
| + | |- | ||
| + | |But, if it is smaller than a hemisphere, and you know this when its depth is less than a half of its surface, measure it as follows: | ||
| + | |style="text-align:right;"|אך אמנם אם היא פחותה מחצי כדור וזה תדעהו כשיהיה עמקה פחות מחצי רחב פיה תמדדה כך | ||
| + | |- | ||
| + | | | ||
| + | *As a pool, whose surface is 6 cubits and one-third, and its depth is 2 cubits. | ||
| + | |style="text-align:right;"|כגון בריכה שרוחב פיה ו' אמות ושליש ועמקה ב' אמות | ||
| + | |- | ||
| + | |First, find its diameter, as I have taught you in the fifth chapter on the measurement of the circular shapes and those that are smaller than a circle. | ||
| + | |style="text-align:right;"|תמצא את קטרה בראשונה כמו שלמדת בפרק החמישי במדידת התמונות העגולות והפחותות מחצי עגולה | ||
| + | |- | ||
| + | | | ||
| + | :When you calculate the diameter of [the sphere formed from] this pool, you find it 7 cubits. | ||
| + | |style="text-align:right;"|וכשתחשוב את קטר הבריכה הזו תמצאנו שהוא ז' אמו‫' | ||
| + | |- | ||
| + | | | ||
| + | :Multiply the 2, which is the depth of the pool, by the 7, which is the diameter of the sphere; it is 14. Multiply it by 3 and one-seventh; it is 44 and this is the area of the pool's surface. | ||
| + | :<math>\scriptstyle{\color{blue}{2\sdot7\sdot\left(3+\frac{1}{7}\right)=14\sdot\left(3+\frac{1}{7}\right)=44}}</math> | ||
| + | |style="text-align:right;"|כפול הב' שהם עמק הבריכה על הז' שהם קטר הכדור יהיו י"ד כפלם ג' פעמים ושביעית פעם יהיו מ"ד והוא מדידת שטח הבריכה | ||
| + | |- | ||
| + | | | ||
| + | :Multiply it by a sixth of the diameter, which is 1 and one-sixth; the result is 51 and one-third and this is the volume of the pool. | ||
| + | :<math>\scriptstyle{\color{blue}{44\sdot\left(\frac{1}{6}\sdot7\right)=44\sdot\left(1+\frac{1}{6}\right)=51+\frac{1}{3}}}</math> | ||
| + | |style="text-align:right;"|כפול אותו בשתות הקטר והוא א' ושתות יעלו נ"א ושליש וזהו תשבורת גוף הבריכה | ||
| + | |- | ||
| + | !<span style=color:Green>Pool larger than a hemisphere</span> | ||
| + | | | ||
| + | |- | ||
| + | |If the pool is larger than a hemisphere, you know this when its depth is greater than a half of its surface: | ||
| + | |style="text-align:right;"|<big>ואם</big> היתה הבריכה יותר מחצי כדור וזה תדעהו כשיהיה העומק יותר ממחצית רחב פיה | ||
| + | |- | ||
| + | | | ||
| + | *As a pool, whose surface is 6 cubits and one-third, and its depth is 5 cubits. | ||
| + | |style="text-align:right;"|כגון בריכה שרחב פיה ו' אמות ושליש ועמקה ה' אמות | ||
| + | |- | ||
| + | | | ||
| + | :When you want to know the diameter of the sphere formed from it, you find it is 7. | ||
| + | |style="text-align:right;"|כשתבקש לדעת קטר הכדור הנחצבת ממנה תמצאנו ז‫' | ||
| + | |- | ||
| + | | | ||
| + | :Multiply the depth, which is 5 cubits, by the 7 cubits of the diameter; the result is 35. Multiply it by 3 and one-seventh; it is 110 and this is the area of the pool's surface. | ||
| + | :<math>\scriptstyle{\color{blue}{5\sdot7\sdot\left(3+\frac{1}{7}\right)=35\sdot\left(3+\frac{1}{7}\right)=110}}</math> | ||
| + | |style="text-align:right;"|תכפול העומק שהם ה' אמות בז' אמות הקטר ההוה ל"ה כפלם ג' פעמים ושביעית פעם יהיו ק"י וזהו מדידת שטח הבריכה | ||
| + | |- | ||
| + | | | ||
| + | :Multiply it by a sixth of the diameter; is 128 and one-third and this is the volume of the pool. | ||
| + | :<math>\scriptstyle{\color{blue}{110\sdot\left(\frac{1}{6}\sdot7\right)=44\sdot\left(1+\frac{1}{6}\right)=128+\frac{1}{3}}}</math> | ||
| + | |style="text-align:right;"|כפול אותה בשתות הקטר יהיו קכ"ח ושליש וזהו תשבורת גוף הבריכה | ||
| + | |- | ||
| + | !<span style=color:Green>Cylinder</span> | ||
| + | | | ||
| + | |- | ||
| + | |If you want to measure a solid called in Greek Kýlindros, which is a long rounded solid, the width of its upper base is equal to [the width of] its bottom base: | ||
| + | |style="text-align:right;"|<big>אם</big> רצית למדוד גוף נקרא בלשון יון קלידירו והוא גוף ארוך מעוגל שוה הרחב ראשו לתושבתו | ||
| + | |- | ||
| + | | | ||
| + | *Example: such a solid whose altitude is 50 cubits, the diameter is 7 cubits, and the perimeter is 22 cubits. | ||
| + | |style="text-align:right;"|דמיון גוף כזה אשר ארכו נ' אמות והקטר ז' אמות והמקיף כ"ב אמות | ||
| + | |- | ||
| + | | | ||
| + | :We find the surface from the perimeter, as we do when measuring the circle; it is 38 cubits and a half. | ||
| + | |style="text-align:right;"|ונמצא מהמקיף כמו שאנו עושים במדידת העגולה התשבורת והוא ל"ח אמות וחצי | ||
| + | |- | ||
| + | | | ||
| + | :Multiply them by the altitude, which is 50; the result is 1925 and it is the volume of this solid. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(38+\frac{1}{2}\right)\sdot50=1925}}</math> | ||
| + | |style="text-align:right;"|כפול אלה על האורך שהם נ' ההוה אלף תתקכ"ה והוא תשבורת הגוף הזה | ||
| + | |- | ||
| + | !<span style=color:Green>Cone</span> | ||
| + | | | ||
| + | |- | ||
| + | |If you want to measure a cone, do as follows: | ||
| + | |style="text-align:right;"|<big>אם</big> רצית למדוד אצטוונא מחודדת תעשה כך | ||
| + | |- | ||
| + | | | ||
| + | *Example: a cone, the diameter of its base is 7 cubits and its altitude from its top is 30 cubits. | ||
| + | |style="text-align:right;"|דמיון אצטוונא שקטר תושבתה ז' אמות והעמוד היורד מראשה ל' אמות | ||
| + | |- | ||
| + | | | ||
| + | :We measure the base by the 7 cubits of the diameter and we find its area, as we do when measuring the circle; the area is 38 cubits and a half. | ||
| + | |style="text-align:right;"|נמדד התושבת מהקטר הז' אמות [ונמצא תשברתה כמו שאנו עושים במדידת העגולה ויהיה התשברת ל"ח אמות וחצי | ||
| + | |- | ||
| + | | | ||
| + | :We take a third of the length of the altitude, which is 10, and multiply it by the 38 and a half; the result is 385 cubits and this is the volume of the mentioned cone. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(\frac{1}{3}\sdot30\right)\sdot\left(38+\frac{1}{2}\right)=10\sdot\left(38+\frac{1}{2}\right)=385}}</math> | ||
| + | |style="text-align:right;"|ונקח מל' אמות]‫<ref>P1031 om.</ref> של גובה העמוד השליש והוא י' ונכפלם על הל"ח וחצי ההוה שפ"ה אמות וזהו תשבורת הגוף של האצטוונא הנזכרת | ||
| + | |- | ||
| + | | | ||
| + | *If you want to find the volume of a cone that has a circular base, whose diameter is 42 cubits, and each lateral side of the cone is 75 cubits. | ||
| + | |style="text-align:right;"|<big>אם</big> רצית למצוא תשבורת אצטוונ' מחודדת ‫<ref>NY 2639 70v</ref>מקשיית ויש לו תושבת העגולה אשר הקטר מ"ב אמות וצלעות גבהות האצטוונא כל אחת‫<ref>Paris end</ref> מע"ה אמות | ||
| + | |- | ||
| + | | | ||
| + | :You want to find the altitude. Find it as follows: | ||
| + | |style="text-align:right;"|ותרצה למצא העמוד תמצאהו כך | ||
| + | |- | ||
| + | | | ||
| + | :Take the length of the lateral side, which is 75 cubits, and multiply them by themselves; the result is 5625. | ||
| + | |style="text-align:right;"|קח גובה הצלעות אשר הם ע"ה אמות וכפלם על עצמם וההווה ה' אלפים תרכ"ה | ||
| + | |- | ||
| + | | | ||
| + | :Half the diameter of the base is 21, multiply it by itself; the result is 441. | ||
| + | |style="text-align:right;"|וחצי קטר התושבת אשר הם כ"א כפלם על עצמם וההווה תמ"א | ||
| + | |- | ||
| + | | | ||
| + | :Subtract it from 5625; 5[184] remains. Extract its root; it is 72 and this is the length of the altitude of the cone. | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{75^2-\left(\frac{1}{2}\sdot42\right)}=\sqrt{75^2-21^2}=\sqrt{5625-441}=\sqrt{5184}=72}}</math> | ||
| + | |style="text-align:right;"|גרעם מה' אלפים תרכ"ה נשארו ה' אלפים קח גדרם הם ע"ב וזהו ארך העמוד של האצטוונה | ||
| + | |- | ||
| + | | | ||
| + | :Find also the area of the circle; it is 1086 cubits. | ||
| + | |style="text-align:right;"|ותמצא גם תשברת העגלה והם אלף פ"ו אמות | ||
| + | |- | ||
| + | | | ||
| + | :Multiply them by a third of the altitude, which is 24; the result is 26064 and this is the volume of the cone. | ||
| + | :<math>\scriptstyle{\color{blue}{1086\sdot\left(\frac{1}{3}\sdot72\right)=1086\sdot24=26064}}</math> | ||
| + | |style="text-align:right;"|כפלם עם שלישית העמוד שהם כ"ד ההווה כ"ו אלף ס"ד וזהו תשברת גוף האצטוונא | ||
| + | |- | ||
| + | | | ||
| + | :If you want to find the area of its surface, take half the diameter of the base, which is 21. Multiply it by the altitude, which is 72; the result is 1512. Multiply it by 22; the result is 33264. Take its seventh; the result is approximately 4755 and this is the area of the surface of the cone. | ||
| + | |style="text-align:right;"|ואם תרצה למצא תשברת שטחה קח חצי קטר התושבת שהם כ"א וכפלם על העמוד שהם ע"ב ההווה אלף תקי"ב כפל אלה על כ"ב ההווה ל"ג אלף רס"ד קח שביעית אלה ההווה ד' אלפים תשנ"ה בקרוב וכן הוא תשברת שטח האצטוונא | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{7}\sdot\left[\left(\frac{1}{2}\sdot42\right)\sdot72\sdot22\right]=\frac{1}{7}\sdot\left(21\sdot72\sdot22\right)=\frac{1}{7}\sdot\left(1512\sdot22\right)=\frac{1}{7}\sdot33264\approx4755}}</math> | ||
| + | |- | ||
| + | !<span style=color:Green>Truncated cone</span> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | *If you want to measure a truncated cone, whose longer diameter is 10 cubits, the shorter is 4 cubits, the altitude is 30 cubits, and you wish to find its volume. | ||
| + | |style="text-align:right;"|<big>אם</big> תרצה למדד אצטוונא מזונבת כלומר חתוכת הראש שהקטר היותר גדול שלה י' אמות והקטן ד' אמות והארך ל' אמות ותרצה למצא תשברת גופה | ||
| + | |- | ||
| + | | | ||
| + | :Do as follows: | ||
| + | |style="text-align:right;"|תעשה כך | ||
| + | |- | ||
| + | | | ||
| + | :Sum up the two diameters, the 10 and the 4; the result is 14. Take its half; it is 7 and we consider it as a diameter. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(10+4\right)\sdot\frac{1}{2}=14\sdot\frac{1}{2}=7}}</math> | ||
| + | |style="text-align:right;"|תחבר שני הקטרים הי' והד' וההווה י"ד וקח חציים והם ז' ונחשבהו קטר | ||
| + | |- | ||
| + | | | ||
| + | :We find the area of the circle, whose diameter is 7, as you know; it is 38 cubits and a half. | ||
| + | |style="text-align:right;"|ונמצא תשברת ‫<ref>71r</ref>העגלה אשר קטרה ז' כמו שידעת ויהיה ל"ח אמות וחצי | ||
| + | |- | ||
| + | | | ||
| + | :Multiply it by the 30 cubits of the altitude; the result is 1155 cubits and this is the volume of the cone. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(38+\frac{1}{2}\right)\sdot30=1155}}</math> | ||
| + | |style="text-align:right;"|תכפלם על הל' אמות של הארך וההווה אלף קנ"ה אמות והוא תשברת גוף האצטוונא | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | === The Fourth Section on the Division of Solids === | ||
| + | |||
| + | |style="width:45%; text-align:right;"|<big>החלק הרביעי</big> בחלוקת הגופניים | ||
| + | |- | ||
| + | |This section is not so necessary in world affairs and bargaining as the previous sections, but very little of it, so we will not turn to explain the division of all the shapes only those that are necessary. | ||
| + | |style="text-align:right;"|זה החלק אינו כל כך צריך בענייני העולם ומקח וממכר כאשר הם החלקים העוברים כי אם מעט מזער ממנו ולכן לא נפנה לפרש חלוקת כל התמונות רק הצריכות לבד | ||
| + | |- | ||
| + | !<span style=color:Green>Cube</span> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | *We say: if you want to divide a cube, whose length is equal to its width and to its height, into two [equal] parts: | ||
| + | |style="text-align:right;"|ונאמר אם תרצה לחלק המעקב והוא אשר ארכו שוה לרחבו ולגבהו לשני חלקים | ||
| + | |- | ||
| + | | | ||
| + | :Draw the diagonal of its base and cut it to half through the diagonal. It is then divided into two [equal] prisms. | ||
| + | |style="text-align:right;"|תוציא אלכסון תושבתו וחתכהו על האלכסון לשנים ויחלק לשני מגוררים | ||
| + | |- | ||
| + | | | ||
| + | *If you want to divide it into three equal parts: | ||
| + | |style="text-align:right;"|ואם רצית לחלקו לשלשה חלקים שוים | ||
| + | |- | ||
| + | | | ||
| + | :Divide the side of its bottom base into three equal parts at two points and likewise the side of the upper base parallel to it, then cut it from one point to one point at right angles. So, it is divided into three equal parts. | ||
| + | |style="text-align:right;"|חלק צלע תושבתו לשלשה חלקים שוים על שתי נקדות וכן צלע הראש הנכחי לו וחתכהו מנקדה אל נקדה על זויות נצבות ויחלק לשלשה חלקים שוים | ||
| + | |- | ||
| + | | | ||
| + | *If you want to divide it into four equal parts: | ||
| + | |style="text-align:right;"|ואם רצית ‫<ref>71v</ref>לחלקו לארבעה חלקים שוים | ||
| + | |- | ||
| + | | | ||
| + | :Divide it first into two [equal] prisms, then draw heights from the [right] angles of the bases to their hypotenuses, so that each of the two prisms is cut in two. By that it is divided into four equal parts. | ||
| + | |style="text-align:right;"|חלקהו בתחלה לשני מגודרים עוד הוציא עמוד מזוית התושבת אל חצי מיתרה ויחלק כל אחד משני המגוררים לשנים ובזה נחלק לארבעה חלקים שוים | ||
| + | |- | ||
| + | | | ||
| + | :Another way: divide the side of the upper base into half and also the side of the bottom base parallel to it. Do the same with the heights. Then, cut them at right angles. By that the cube is divided into four equal parts. | ||
| + | |style="text-align:right;"|ובאופן אחר חלק צלע הראש לשנים וכן צלע התושבת הנכחי לו גם ככה תעשה בצלעות הגובה וחתכם על זויות נצבות ובזה נחלק המעקב לארבעה חלקים שוים | ||
| + | |- | ||
| + | !<span style=color:Green>Prism whose base is a rectangle</span> | ||
| + | | | ||
| + | |- | ||
| + | |If it is a rectangle and its altitude at a right angle is different from its length and breadth, or equal to them, its division is as the division of the cube. | ||
| + | |style="text-align:right;"|ואם היה מרבע ארוך נכחי הצלעות נצב הזויות וגבהו על זויות נצבות מתחלף לארכו ורחבו או שוה לו חלוקתו בחלוקת המעוקב | ||
| + | |- | ||
| + | ! | ||
| + | <span style=color:Green>Prism whose base is a rhombus</span> | ||
| + | | | ||
| + | |- | ||
| + | |If it is a rhombus, its division into two is by that you cut it at the diagonal of its bottom base that extends from vertex to vertex. | ||
| + | |style="text-align:right;"|ואם היה מעויין חלוקתו לשנים הוא שתחתכנו על האלכסון של תושבתו היוצאת מן הזוית אל הזוית | ||
| + | |- | ||
| + | |There is no difference whether the diagonal is drawn from an acute angle to an acute angle, or from an obtuse angle to an obtuse angle. | ||
| + | |style="text-align:right;"|ואין הפרש בין אם יצא האלכסון מזוית החדה אל החדה או מן הנרחבת אל הנרחבת | ||
| + | |- | ||
| + | |If you divide it into three, divide it through the sides, as you did with the cube. | ||
| + | |style="text-align:right;"|ואם תחלקנו לשלשה חלקהו מהצלעות כמו שעשית במעקב | ||
| + | |- | ||
| + | |If into four, divide it through the two diagonals, as you did with the cube, or by the other way mentioned there. | ||
| + | |style="text-align:right;"|ואם לארבעה תחלקנו על ידי שני האלכסונים כמו שעשית במעקב או באופן האחר הנזכר שם | ||
| + | |- | ||
| + | |So is the rule of division of the parallelogram. | ||
| + | |style="text-align:right;"|וכן משפט חלוקת הדומה למעויין | ||
| + | |- | ||
| + | !<span style=color:Green>Prism whose base is triangular</span> | ||
| + | | | ||
| + | |- | ||
| + | |If you wish to divide the prism, whose bottom base is triangular, whose upper base is triangular, and whose faces are quadrangular, into two [equal] parts: | ||
| + | |style="text-align:right;"|ואם רצית לחלק המגורר שתושבתו משלשת וראשו משלש וצלעות גבהו מרבעים לשני חלקים | ||
| + | |- | ||
| + | |Draw an altitude from a vertex of its bottom base to the side parallel to it that is opposite to this vertex and divide it through the altitude at right angle, so the prism is divided into two equal parts. | ||
| + | |style="text-align:right;"|הוצא מן זוית תושבתו אל הצלע הנכחי לו אשר הוא מיתר לזאת הזוית עמוד וחלקהו על העמוד על זויות נצבות וכבר נחלק המגורר לשני חלקים שוים | ||
| + | |- | ||
| + | |If one of the sides of the base is longer than the other sides, draw the altitude from the vertex that is opposite to that side and divide it through it. | ||
| + | |style="text-align:right;"|ואם הצלע האחד מצלעות התושבת ארוך משאר הצלעות הוצא עליו העמוד מהזוית שכנגד הצלע הזה וחלקהו עליו | ||
| + | |- | ||
| + | |In another way: divide the lateral sides in two and cut it through there. | ||
| + | |style="text-align:right;"|ובאופן אחר חלק צלעות הגבהות לשנים וחלקהו משם | ||
| + | |- | ||
| + | |If you wish to divide it into three [equal] parts, divide it into three pyramids and this is explained in Euclid's XII. | ||
| + | |style="text-align:right;"|ואם רצית לחלקו בשלשה חלקים חלקהו שלשה מחדדים וזה התבאר בי"ב לאקלידס | ||
| + | |- | ||
| + | !<span style=color:Green>Sphere</span> | ||
| + | | | ||
| + | |- | ||
| + | |If you want to divide the surface of the sphere do as follows: | ||
| + | |style="text-align:right;"|‫<ref>72r</ref>ואם רצית לחלק הכדור בשטחו תעשה כך | ||
| + | |- | ||
| + | |Mark on it two opposite points that are farthest from each other of all the points that can be on the sphere and they are called the poles of the sphere. | ||
| + | |style="text-align:right;"|סמן עליו שתי נקדות מקבילות היותר רחוקות זו מזו מכל הנקדות שאפשר ליפול בכדור והם יקראו קטבי הכדור | ||
| + | |- | ||
| + | |Make one of the two points a center and draw a circle about half the distance between it and the other point, so they are the larger and middle circles. | ||
| + | |style="text-align:right;"|ועשה נקדה אחת משתיהן מרכז וסבב עגלה כמרחק מחצית המרחק אשר בינה ובין הנקדה האחרת והיא העגלה היותר גדולה והאמצעית | ||
| + | |- | ||
| + | |Divide this circle again according to the number of parts, into which you want to divide the surface of the sphere, by marking points or other signs. | ||
| + | |style="text-align:right;"|עוד חלק העגלה הזאת לפי כמות החלקים שתרצה לחלוק שטח הכדור על ידי סימנים נקודות או זולתם | ||
| + | |- | ||
| + | |Then, draw semicircles, each of which pass through the two poles of the sphere and through the points that are on the middle circle. | ||
| + | |style="text-align:right;"|אחר כן תעגל חציי עגולות שתעברנה כל אחת על שתי קטבי הכדור ועל הנקדות שהם בעגלה האמצעי‫' | ||
| + | |- | ||
| + | |By that the surface of the sphere is divided as you wish. | ||
| + | |style="text-align:right;"|ובזה יחלק שטח הכדור לפי רצונך | ||
| + | |- | ||
| + | |Do the same if you want to divide a hemisphere, or [a part of a spherical solid] greater than a hemisphere. | ||
| + | |style="text-align:right;"|וככה תעשה אם תרצה לחלק חצי כדור או יותר מחציו | ||
| + | |- | ||
| + | |So, the circles pass through the pole and the circumference of the semicircle that replaces the middle circle of the sphere, or the circumference of the part that is greater than a hemisphere. | ||
| + | |style="text-align:right;"|ואז תעברנה העגולות על הקטב ועל שפת חצי העגלה שהוא במקום העגלה האמצעית של הכדור או על שפת החתיכה הגדולה מחצי הכדור | ||
| + | |- | ||
| + | |Likewise if the part [of a spherical solid] is smaller than a hemisphere. | ||
| + | |style="text-align:right;"|גם ככה אם החתיכה קטנה מחצי ‫<ref>72v</ref>הכדור | ||
| + | |- | ||
| + | |All the divisions we have mentioned have proofs in geometry, but we did not bring them, because they are not of great need for what we intended to explain. | ||
| + | |style="text-align:right;"|ובכל אלה החלוקות שהזכרנו יש מופתים בחכמת המדות ולא הבאנו אותם כי לא היה צרך גדול במה שכווננו לבאר | ||
| + | |- | ||
| + | |I have taught you everything you need to know in this science. | ||
| + | |style="text-align:right;"|הנה הודעתיך כל מה שצריך לדעת בזאת המלאכה | ||
| + | |- | ||
| + | |To God alone be the glory and praise forever Amen. | ||
| + | |style="text-align:right;"|ולאל לבדו התהלה והשבח לעולמים אמן | ||
| + | |- | ||
| + | |Over and done. | ||
| + | |style="text-align:right;"|תם ונשלם‫<ref>NY 2639: נשלם הספר חכמת המספר וחכמת המדות שחבר מרנו ורבנו החכם ב"ר מרדכי כומטיינו יצ"ו בהכ"ר אליעזר כומטיינו יעמ"ש ונשלם ע"י לי שבתי בכ"ר משה ז"ל מקנדייה שנת הרל"ח בכ"ד לחדש אדר שבח לאל יתעלה אשר לו התהלה והיכולת וזאת היא ההעתקה השנית וכתבתיהו לר' כלב המכונה אפדופילו י"ל בכ"ר אליהו יצ"ו בכ"ר יהודה</ref> | ||
|} | |} | ||
| Line 7,529: | Line 10,984: | ||
::[[http://www.bl.uk/manuscripts/Viewer.aspx?ref=add_ms_27107_fs001r London27107]] | ::[[http://www.bl.uk/manuscripts/Viewer.aspx?ref=add_ms_27107_fs001r London27107]] | ||
:3) New York, Jewish Theological Seminary Ms. 2616 (IMHM: f 28869), (19th century) (Book 2) | :3) New York, Jewish Theological Seminary Ms. 2616 (IMHM: f 28869), (19th century) (Book 2) | ||
| − | ::[[ | + | ::[[https://www.nli.org.il/he/discover/manuscripts/hebrew-manuscripts/viewerpage?vid=MANUSCRIPTS&docid=PNX_MANUSCRIPTS990001053530205171-1#$FL30378174 NY2616]] |
:4) New York, Jewish Theological Seminary Ms. 2632 (IMHM: f 28885), (19th-20th century)<br> | :4) New York, Jewish Theological Seminary Ms. 2632 (IMHM: f 28885), (19th-20th century)<br> | ||
::[[http://web.nli.org.il/sites/NLI/Hebrew/digitallibrary/pages/viewer.aspx?presentorid=MANUSCRIPTS&docid=PNX_MANUSCRIPTS000105384-1#|FL30377181 NY2632]] | ::[[http://web.nli.org.il/sites/NLI/Hebrew/digitallibrary/pages/viewer.aspx?presentorid=MANUSCRIPTS&docid=PNX_MANUSCRIPTS000105384-1#|FL30377181 NY2632]] | ||
:5) New York, Jewish Theological Seminary Ms. 2633 (IMHM: f 28886), (16th century) | :5) New York, Jewish Theological Seminary Ms. 2633 (IMHM: f 28886), (16th century) | ||
| − | ::[[ | + | ::[[https://www.nli.org.il/he/discover/manuscripts/hebrew-manuscripts/viewerpage?vid=MANUSCRIPTS&docid=PNX_MANUSCRIPTS990001053850205171-1#$FL30379096 NY2633]] |
:6) New York, Jewish Theological Seminary Ms. 2639 (IMHM: f 28892), (1478) | :6) New York, Jewish Theological Seminary Ms. 2639 (IMHM: f 28892), (1478) | ||
| − | ::[[ | + | ::[[https://www.nli.org.il/he/discover/manuscripts/hebrew-manuscripts/viewerpage?vid=MANUSCRIPTS&docid=PNX_MANUSCRIPTS990001058500205171-1#$FL30365208 NY2639]] |
:7) Oxford, Bodleian Library MS Heb. d. 5/1 (IMHM: f 22729), ff. 1r-17r (Cat. Neub. 2774, 1); (1522) | :7) Oxford, Bodleian Library MS Heb. d. 5/1 (IMHM: f 22729), ff. 1r-17r (Cat. Neub. 2774, 1); (1522) | ||
:8) Paris, Bibliothèque Nationale de France heb. 1031/3 (IMHM: f 15723), ff. 26r-64v (15th-16th century)<br> | :8) Paris, Bibliothèque Nationale de France heb. 1031/3 (IMHM: f 15723), ff. 26r-64v (15th-16th century)<br> | ||
::[[https://gallica.bnf.fr/ark:/12148/btv1b90644811/f27.image.r=Hébreu.langEN Paris 1031]] | ::[[https://gallica.bnf.fr/ark:/12148/btv1b90644811/f27.image.r=Hébreu.langEN Paris 1031]] | ||
:9) St. Petersburg, Russian National Library Evr. I 320b (IMHM: f 50984) (1495) | :9) St. Petersburg, Russian National Library Evr. I 320b (IMHM: f 50984) (1495) | ||
| − | ::[[ | + | ::[[https://www.nli.org.il/he/discover/manuscripts/hebrew-manuscripts/viewerpage?vid=MANUSCRIPTS&docid=PNX_MANUSCRIPTS990000834490205171-1#$FL37945783 Evr. I 320]] |
:10) St. Petersburg, Russian National Library Evr. I 343-344 (IMHM: f 50961, f 41596, f 41597) (Istanbul, 1485) | :10) St. Petersburg, Russian National Library Evr. I 343-344 (IMHM: f 50961, f 41596, f 41597) (Istanbul, 1485) | ||
| − | ::[[ | + | ::[[https://www.nli.org.il/he/discover/manuscripts/hebrew-manuscripts/viewerpage?vid=MANUSCRIPTS&docid=PNX_MANUSCRIPTS990001513920205171-1#$FL38597400 Evr. I 343-344]]<br> |
<span style=color:blue>The transcript of the second book is based mainly on manuscript Paris 1031</span> | <span style=color:blue>The transcript of the second book is based mainly on manuscript Paris 1031</span> | ||
Latest revision as of 12:03, 15 December 2022
Contents
- 1 Introduction
- 2 Book One
- 3 The Second Book of this Treatise Discusses Geometry
- 3.1 [The First Section]
- 3.1.1 Chapter One of the First Section of the Second Book
- 3.1.2 Chapter Two [on the Measuring of the Various Quadrangles]
- 3.1.3 Chapter Three on the Measuring of the Various Triangles
- 3.1.4 Chapter Four on the Measuring of the Various Trapezoids
- 3.1.5 Chapter Five on Knowing the Round Shapes
- 3.1.6 Chapter Six on the Measuring of Regular Polygons
- 3.2 [The Second Section on the Division of Plane Shapes]
- 3.3 The Third Section on the Measuring of Solids
- 3.4 The Fourth Section on the Division of Solids
- 3.1 [The First Section]
- 4 Notes
- 5 Apparatus
- 6 Appendix I: Glossary of Terms
- 7 Appendix II: Bibliography
Introduction |
|
| Said Mordecai the son of his honor the rabbi Eliezer Comṭino may he rest in peace from Constantinople: | [1]אמר מרדכי בכ"ר אליעזר כומטיינו יעמ"ש הקושטנדיני |
| Since the existences were emanated from the first principle of all gradually and orderly, step by step, we find each having the perfection it deserves according to its final cause, and we find the definition of each. | להיות שההתחלה הראשונה לכל הושפעו ממנה שאר הנמצאות במדרגה ובסדר מדרגה אחר מדרגה ובזה נמצא כל אחד לפי שלמותו הראוי לו עד תכליתו ונמצא לכל דבר חקו |
| This perfection clings to its owner constantly, does not depart from it. | והשלמות הזה רודף לבעליו על התמידות בלתי סר ממנו |
| This with reference to things whose existence does not depend on our actions. | וזה בדברים אשר אין מציאותם בפעולותינו |
| When we want the things, whose existence depends on our actions, to be perfect, we compare them to the things whose existence does not depend on our actions, according to our ability to compare them to things whose existence does not depend on our actions. | [והדברים אם מציאותם בפעולותנו][2] כאשר רצינו להיותם שלמים נמשילם אל הדברים אשר אין מציאותם בפעולותינו כפי היכולת ראוי לנו ג"כ אם רצינו השלימות והסדור לדמות הדברים אשר מציאותם בפעולותינו [לזה כפי היכולת][3] |
| The things whose existence depends on our actions are divided into two types: | [והדברים אשר מציאותם בפעולותנו][4] יחלקו לשני סוגים |
|
האחד הדברים שבין האדם לעצמו |
|
והשני הדברים שבין האדם לזולתו |
| The first type is not included in this treatise, although the philosophers wrote many books about it also. | והסוג הראשון בלתי נכנס בחבור הזה ואע"פ שגם בזה חברו הפלוסופים ספרים הרבה |
| For the purpose of this treatise is in what happens from him to others. | כי כונת זה החבור הוא במה שיפול ממנו אל זולתו |
| Although the perfection that occurs in things, whose existence does not depend on our actions, is from one essence to another, it does not return to it, but proceed straightly. | ואם השלמות הנופלת בדברים אשר אין מציאותם בפעולותינו מהעצם האחד לזולתו בלתי חוזרת אליו אבל הולכת על יושר |
| While the perfection that occurs in things whose existence depends on our actions is circular. | והשלמות אשר תפול בדברים אשר מציאותם בפעולותינו תלך על דרך הסבוב |
| The resemblance is that [both perfections are] from one to one, from the aspect of what is from and to. | הדמיון יהיה במה שממנו לאליו מצד מה שהוא ממנו ואליו |
| This type is divided into three kinds: | וזה הסוג יחלק לשלשה חלקים |
|
האחד הדברים אשר יגיעו מהאיש לזולתו על דרך החמלה והחנינה |
|
והשני הדברים אשר יגיעו לו על צד הצדק והיושר |
|
והשלישי הוא מהדברים אשר יגיעו מהאדם לזולתו על צד העול והחמס |
| The first kind are the things that exceed equality. | והחלק הראשון הוא הדבר המוסיף על השווי |
| The third kind are things that reduce equality. | והחלק השלישי הוא דבר גורע מהשווי |
| The second is equality itself. | והשני הוא השווי בעצמו |
| Hence, the second is the root and foundation of the [two] other kinds and the investigation should concentrate on it. | א"כ יהיה השני שרש ויסוד לשאר החלקים ולכן ראוי שתהיה החקירה בו |
| As I saw that this kind is found in two aspects: | ובראותי שזה החלק משתמש לשני סוגים |
|
הסוג האחד במקח וממכר וזהו הנקרא בלשון חכמינו ז"ל אונאה אם היה שם שתות הפרש בין הצדק ובין המכר וזה הדבר הוא בלתי מוגבל ולכן לא תקיף בו ידיעה כי ישתנו מחירי הדברים בכל זמן מפני זה לא נדבר בו |
|
והסוג השני הוא בחשבון ובמדות כי אחר שנתפשרו שני הצדדים בקנין א' כלל אחד בכך וכך מחיר בחלקו המונה אותו אחר כשיבאו לכפול המחיר כמנין החלקים אשר נמנה בהם הכלל ולא יצא החשבון שוה להעדר ידיעתם בחשבון או במדידת הקרקעות יהיה מזה עול וחמס ויוסר הצדק משם ותחת אשר נרצה להדמות אל הדברים אשר אין מציאותם בפעולותינו נתרחק מהם הרבה ואוי על הקנין הרע הזה אשר נקנה לנפשינו |
| Therefore, I decided to compose this book in order to remove injustice from human beings and so justice will prevail among them. | לכן ראיתי לחבר זה הספר כדי שאסיר זה העול מבני אדם ויהיה הצדק נמצא ביניהם |
| I divided it into two books: | וחלקתי אותו לשני ספרים |
|
הספר הראשון מדבר בעניני החשבון |
|
והספר השני מדבר בעניני המדות |
| I have preceded the book on arithmetic to the book on geometry, because arithmetic precedes by nature to the other three mathematical sciences, as Nicomachus of Gerasa explained in his Introduction to Arithmetic. | והקדמתי [5]ספר החשבון לספר המדות כי חכמת החשבון קודמת בטבע לשאר מיני ההרגליות השלש כאשר באר זה ניקומאכוש [הגהרשני][6] בהצעת מאמרו מספר האירתימיטיקא |
| I divided the first book into four sections: | והספר הראשון חלקתי אותו אל ארבעה חלקים |
|
הראשון לקבץ מספר עם מספר ולחסר מספר ממספר |
|
והשני לכפול מספר על מספר |
|
[והשלישי לחלק מספר על מספר][7] ולהוסיף מספר על מספר |
|
והרביעי לערך מספר על מספר |
|
עוד שמתי חלק אחד אחריהם לדבר על הנשברים |
| By this I followed the practice of the Christians to speak briefly so as not to confuse the student. | ודרכתי בזה הדרך דרך הנוצרים לדבר בקיצור כדי שלא יתבלבל התלמיד |
| I divided the second book also into four sections: | עוד הספר השני חלקתי אותו לארבעה חלקים |
|
האחד במדידת התמונות השטחיות כגון העגולות והמשולשים והמרובעים וזולתם |
|
והשני בחלוקתם |
|
והשלישי במדידת התמונות הגופניות |
|
והרביעי בחלוקתם |
| Here I start with the help of the One who grants knowledge to man [Psalms 94, 10]. | ומהנה אתחיל בעזרת המלמד לאדם דעת[note 1] |
Book OnePart One: Addition & Subtraction |
|||||||||||||||||||||||||||
Chapter One of The First Part of The First Book: Introduction |
הפרק הראשון מהחלק הראשון מהספר הראשון | ||||||||||||||||||||||||||
| We should inform why we precede [the discussion on] the addition of a number to a number before the rest of the operations. | ראוי שנודיע למה הקדמנו קבוץ מספר על מספר משאר החלקים | ||||||||||||||||||||||||||
| I say that it is because it is simpler than them and it precedes them by nature, since it is simpler than them, as each of them consists of it and of the property that is specific for it; and for any thing that is composed of a thing, the thing of which it is composed is simpler than the one that is composed. | ואומר לפי שהוא יותר פשוט מהם וקודם בטבע מהם אם היותו יותר פשוט מהם להיות שכל אחד מהם מורכב מזה ומהענין המיוחד לו והדבר אשר יורכב מדבר הדבר אשר יורכב ממנו הוא יותר פשוט מהמורכב ההוא | ||||||||||||||||||||||||||
| I say that [each operation] consists of it, I mean, of one of its two categories or of both together. | [ואומר][8] שכל אחד מורכב מזה ארצה מאחד משני חלקיו או משניהם יחד | ||||||||||||||||||||||||||
| Because it is divided into two types: | כי זה יחלק לשני מינים | ||||||||||||||||||||||||||
|
אם שיהיה הקבוץ הזה בלתי משתנה ממדרגת נקבציו וזה באחד עד תשעה פעמים ובשנים עד ארבעה פעמים ובשלשה עד שלשה פעמים ואם יורכב מאלה [יהיה][9] לפי חשבונו המיוחד לו | ||||||||||||||||||||||||||
|
או שיהיה הקבוץ הזה משתנה ממדרגת נקבציו וזה ישתנה ויחלק לשני חלקים | ||||||||||||||||||||||||||
|
אם שישתנה ממדרגת נקבציו ולא יהיה שם רושם כלל מהמדרגה ההיא | ||||||||||||||||||||||||||
|
או שישתנה וישאר גם רושם מדרגת נקבציו שם | ||||||||||||||||||||||||||
| Therefore it precedes those that consist of it. | ולכן הוא קודם מהמורכבים ממנו | ||||||||||||||||||||||||||
| One cannot say that because it is simple, it should follow the composite, according to the Aristotle's explanation at the beginning of the Physics that the general should precede the special, for three reasons: | ואין לאומר שיאמר כי להיותו פשוט ראוי לאחרו מן המורכב ממה שבאר ארסטו בתחלת ספר השמע שראוי להקדים הכולל מהמיוחד לשלש סבות | ||||||||||||||||||||||||||
|
האחת שהכולל יותר ידוע מהמיוחד וראוי ללכת מהיותר ידוע אל היותר מוסכל | ||||||||||||||||||||||||||
|
והשנית שלא נשיב המאמר האחד פעמים הרבה | ||||||||||||||||||||||||||
|
והשלישית שתהיינה ההקדמות מיוחדות כי יצדק הכולל שגדרו נשוא על המיוחד כאשר ינשא גדר המין על אישיו וכן המספר נשוא על חלקיו | ||||||||||||||||||||||||||
| But, the definition of ten from the aspect of its being a ten, is not superior to any other number, not to nine and not to any other. | אולם גדר העשרה מצד מה שהם עשרה לא ינשאו על מספר אחר לא על [10]תשעה ולא על זולתו | ||||||||||||||||||||||||||
| Therefore, the special should precede the general, because the general is known from its summing. | ולכן בכזה ראוי להקדים המיוחד על הכולל כי מקבוצו יודע הכולל | ||||||||||||||||||||||||||
| It precedes them by nature, since when it is conceived they are conceived also, but not vice versa, and when they are found it is found also. | ואם היותו קודם בטבע מהם מפני [שכאשר][11] נעלה הוא יעלו גם הם ולא יתהפך וכשימצאו הם ימצא גם הוא | ||||||||||||||||||||||||||
| For these reasons, it should precede them. | ומפני אלו הסבות היה ראוי להקדימו מהם | ||||||||||||||||||||||||||
| We should note first that all the numbers are included in nine. | וראוי שנקדים להזכיר קודם שכל המספרים נכללים בתשעה | ||||||||||||||||||||||||||
| Since from one to nine the units are completed; and as you arrive to the ten you have reached one tithe [= one unit of the rank of tens], until you complete the tens when arriving to ninety; again from a hundred you start with a unit [of the rank of hundreds] and when arriving to nine hundreds you have completed the hundreds; until you start once more from a thousand as a unit [of the rank of thousands]; and so on endlessly. | כי מאחד עד תשעה ישלמו האחדים ובהגיעך אל העשרה הגעת אל עשירית אחת עד שתשלים [כל][12] העשרות בהגיעך עד תשעים עוד ממאה התחלת באחד ובהגיעך אל תשע מאות השלמת כל המאות עד שתתחיל עוד מאלף אחד וכן ללא תכלית | ||||||||||||||||||||||||||
| Be it by nature, as many have thought, or by free choice, as we think, now it is not the time to decide on that, for this is not our intention | ולהיות זה בטבע כאשר חשבו רבים או בבחירה כאשר נחשב אנחנו אין עת [להכריע][13] עתה בזה כי אינו מכונתינו | ||||||||||||||||||||||||||
| Therefore the people of India have applied nine letters as being indicators of nine numbers; and the diversity of their ranks indicates the diversity of the ranks of ninths, as we will explain. | ולכן תקנו אנשי הודו תשעה אותיות להיותן מורין על תשעה מספרים והתחלפות מדרגותם יורו על התחלפות מדרגות התשיעיות כאשר נבאר | ||||||||||||||||||||||||||
| These are their shapes: | וזה צורתם | ||||||||||||||||||||||||||
| 1 2 3 4 5 6 7 8 9. | 1 2 3 4 5 6 7 8 9 | ||||||||||||||||||||||||||
| They were not satisfied with the letters of the alphabet, as each of them has a special accepted shape, which is not so for those letters, since they are devoid of all attributes, shared by every shape applied on them, they are as the form of the prime matter, and their positional rank is the same as their shape [= that is to say, their shape does not change when placed in different ranks]. | ולא הסתפקו עם אותיות האלפבית כי לכל אחד מהם צורה מיוחדת הנחיית מה שאין כן לאלו האותיות כי הן מופשטו' מכל הנחה ומשותפות לכל צורה שתחול עליהן והן כדמות ההיולי הראשון ומדרגת הנחתן היה כמו הצורה להן | ||||||||||||||||||||||||||
| So every person can create other letters different than those. | וכן יוכל האדם לחדש אותיות אחרות זולת אלה | ||||||||||||||||||||||||||
| By saying letters I do not mean the letters of the alphabet, but signs devoid of any numerical [value] as these, although all arithmeticians already tend to use these. | ואמרי אותיות לא ארצה מן אותיות האלפביתא אבל סימנים מופשטים מכל חשבון כאלה אבל כבר נהגו כל בעלי החשבון להשתמש באלה | ||||||||||||||||||||||||||
Chapter Two: Addition |
הפרק השני | ||||||||||||||||||||||||||
| It should be noted that the numbers, which are from one to nine, are called "units"; and from the tens onwards they called "kelalim" [i.e. products of ten], namely the tens, hundreds, thousands, tens of thousands and the rest. | ראוי להודיע כי המספרים אשר הם מאחד עד תשעה יקראו פרטים ומן העשרות ומעלה יקראו כללים ר"ל העשרות והמאות והאלפים והרבבות והשאר | ||||||||||||||||||||||||||
| Though they are called by the name "kelal", their rank is not one; there is only one kelal which includes another kelal and so on until reaching the units. | ואע"פ שיקראו בשם הכלל אבל אין מדרגתם אחת רק יש כלל כולל כלל אחד וכן זה אחד עד שיגיע אל האחדות | ||||||||||||||||||||||||||
| Therefore, the arithmeticians should write the ranks of their number orderly by writing the most inclusive kelal first and after it the lower kelal and so on successively until reaching the units. | לכן ראוי לבעלי החשבון לכתוב [מדרגות][14] חשבונם כסדר שיכתבו הכלל היותר כולל ראשונה ואחריו הכלל השפל ממנו וכן על הסדר עד שיגיעו אל האחדים | ||||||||||||||||||||||||||
| When they turn to write another number beneath them, they should keep this order and write each kelal under its corresponding, i.e. the tens of thousands under the tens of thousands, the thousands under the thousands, the hundreds under the hunderds, and the units under the units. | עוד כשיבאו לכתוב מספר אחר תחת אלה שישמרו הסדר הזה ויכתבו כל כלל תחת הדומה לו ר"ל הרבבה תחת הרבבה והאלף תחת האלפים והמאה תחת המאות והאחדים תחת האחדים | ||||||||||||||||||||||||||
| So, we call the units "first rank", the tens "second rank", the hundreds "third rank", the thousands "furth rank" and so on endlessly. | ונקרא א"כ האחדים מדרגה ראשונה והעשרות מדרגה שניה והמאות מדרגה שלישית והאלפים מדרגה רביעית וכן ללא תכלית | ||||||||||||||||||||||||||
| If you have a number that is not arranged in all its ranks, as when you have thousands, tens, and units, yet the hundreds are missing there. Be careful not to write the tens next to the thousand, but write the thousands and in the place of the hundreds write a zero [lit. circle], 0, called in Arabic "sifra", in Greek "oudến" and in foreign language "nulla"; meaning that here is the place of the hundreds and there is nothing there. | ואם יהיה בידך מספר שאינו מסודר בכל מדרגותיו כגון שהיו בידך אלפים ועשרות ואחדים והנה המאות הם חסרים משם השמר לך שלא תכתוב העשרות בצד האלפים אבל תכתוב האלפים ובמקום המאות תכתוב גלגל א' 0 הנקרא בלשון [15]ישמעאל סיפרא ובלשון יון אודין ובלשון לעז נולא כלומר כי בכאן הוא מקום המאות ואין שם דבר | ||||||||||||||||||||||||||
| After the zero, write the tens in their place, and by that the ranks will be together one after the other in a straight line. | ואחר הגלגל תכתוב העשרות במקומו ובזה יהיו המדרגות כל אחת עם חברתה על קו ישר | ||||||||||||||||||||||||||
| When you want to sum up the lines making them one number, always begin from the units and go down in a straight line to the units below them in all the lines. | וכשתרצה לקבץ כל הטורים לעשות אותם סך אחד תעשה ותתחיל מן האחדים לעולם ותרד על קו ישר באחדים אשר תחתיהם בכל הטורים | ||||||||||||||||||||||||||
| If they do not reach ten, write what you find beneath all, corresponding to the units below. | ואם לא יעלו עד עשר כתוב מה שתמצא תחת הכל כנגד האחדים למטה | ||||||||||||||||||||||||||
| If they are more than ten, write in the [rank of] tens a dot for each ten, i.e. as the number of tens, so is the [number of] dots you write, and write what remains that does not reach ten beneath the units. | ואם יעלו יותר מעשר בכל עשירית ועשירית כתוב נקדה אחת בעשרות ר"ל כמספר העשרות יהיו הנקדות שתכתוב והנשארים אשר לא הגיעו לכלל עשר כתוב אותם תחת האחדים | ||||||||||||||||||||||||||
| Go down the rank of tens also and sum them up together with the dots you wrote. | עוד תרד במדרגת העשרות ועם הנקדות שכתבת וקבצם | ||||||||||||||||||||||||||
| Proceed according to the rule that if you do not get ten, write what you find beneath the tens; if you get tens, write dots in the [rank of] hundreds as the number of the resulting tens, and write what remains that does not reach ten beneath the rank of tens. | ותעשה כמשפט הזה כי אם לא תגיע לעשר כתוב מה שתמצא תחת העשרות ואם הגעת לעשרות ככמות העשרות שעלו תעשה נקדות במאות והשאר אשר לא הגיעו לעשרות כתבם תחת מדרגת העשרות | ||||||||||||||||||||||||||
| And so on endlessly. | וכן אל בלתי תכלית | ||||||||||||||||||||||||||
| The total sum beneath in each of the ranks is less than ten; what is in the rank of units of the total sum are units; in the rank of the tens are tens and so on in each rank according to its position. | ואם כן יהיה הסך למטה בכל אחת מהמדרגות פחות מעשר והנמצאים בסך במדרגת האחדים הם אחדים ובמדרגת העשרות הם עשרות וכן בכל מדרגה כפי מדרגתה | ||||||||||||||||||||||||||
| If the lines you want to sum are very many, divide them into three parts, or four, or less, or more, according to the number of the lines. Do with each part by itself what was shown, then gather the total sums of all the parts and sum them up to one sum according to this same rule; the sum is the total sum of all parts together. | ואם היו הטורים אשר רצית לעשות אותם סך הרבה מאד תחלקם לשלשה חלקים או לארבעה או לפחות או ליתר לפי רוב הטורים ומיעוטם ותעשה סך לכל חלק וחלק בפני עצמו כאשר הראית אחר כן תחבר סכי כל החלקים ותעשה אותם סך כזה המשפט בעצמו והמקובץ יהיה סך כל החלקים יחד | ||||||||||||||||||||||||||
| This is the type of addition called sum: | וזאת צורת הקבוץ הנקרא סך | ||||||||||||||||||||||||||
|
רצינו לקבץ אלף ומאתים וחמש עשרה ואלפים ושלש מאות ועשרים ושנים | ||||||||||||||||||||||||||
|
כתבנו אותם סך כך | ||||||||||||||||||||||||||
| |||||||||||||||||||||||||||
|
אחר חברנו אותם והיה קבוצם למטה שבע אחדים ושלש עשרות וחמש מאות ושלשת אלפים וידענו שהם שלשת אלפים וחמש מאות ושלשים ושבעה | ||||||||||||||||||||||||||
|
ואלה המספרים בסכום היורד לא הגיעו לכלל עשר בשום מדרגה | ||||||||||||||||||||||||||
|
ונצייר עוד צורה אחרת המגעת במדרגותיה לכלל העשרות והיא שנחבר עוד תחת אלה השני טורים שני טורים אחרים האחד תשעת אלפי' ושש מאות וחמשים ושבעה והשנית שמנת אלפים וחמש מאות וששים ושלש | ||||||||||||||||||||||||||
|
וזאת היא הצורה | ||||||||||||||||||||||||||
| |||||||||||||||||||||||||||
|
קבצנו אותם ויצא הסך למטה שבעה אחדים וחמש עשרות ושבע מאות ואלף אחת ושתי רבבות | ||||||||||||||||||||||||||
|
והנה קבוץ האחדים כאשר תרד במדרגותם על קו היושר תמצאם שבעה עשר הוצאנו העשרה מהם וכתבנו נקדה אחת במדרגת העשרות ונשארו שבעה וכתבנו אותם תחת האחדים | ||||||||||||||||||||||||||
|
עוד כאשר קבצנו מדרגת העשרות על קו היורד ביושר עם הנקדה שכתבנו [16]שם בעד העשירית שהוצאנו מהאחדים מצאנום חמש עשרה הוצאנו עוד העשרה מהם וכתבנו נקדה בעבורם במדרגת המאות והחמשה הנשארים כתבנום למטה במדרגתן של העשרות | ||||||||||||||||||||||||||
|
וכן כשקבצנו המאות בירידתנו על קו היושר יחד עם הנקדה שכתבנו כנגד העשירית שהוצאנו מהעשרות מצאנום שבעה עשר הוצאנו מהם העשרה וכתבנו בעדם נקדה במדרגת האלפים והשבעה הנשארים כתבנו אותם במדרגת המאות | ||||||||||||||||||||||||||
|
וכן כשקבצנו את האלפים בירידתנו על קו היושר עם הנקדה שכתבנו עמם בעד העשירית שהוצאנו מהמאות מצאנו אותם עשרים ואחד הוצאנו העשרים שהם ב' עשרות וכתבנו בעבורם שתי נקדות במדרגת הרבבות והאחד הנשאר כתבנוהו תחת האלפים | ||||||||||||||||||||||||||
|
וידענו מזה שהם שתי רבבות ואלף אחד ושבע מאות וחמשים ושבעה | ||||||||||||||||||||||||||
Check |
|||||||||||||||||||||||||||
|
מאזנים לדעת אם הסכום שלך אפשר להיותו אמת על דרך התשעה | ||||||||||||||||||||||||||
|
תחשוב כל המדרגו' כאלו הם אחדים ותקבצם ותשליכם תשעה תשעה ומה שישאר שמרהו ואם לא ישאר דבר תחשוב בלבך סיפרא | ||||||||||||||||||||||||||
|
עוד קבץ מדרגות הסך גם הוא כאלו הם אחדים והשליכם ט' ט' ואם ישארו בידך ההשארות כראשון אפשר שחשבונך יהיה אמת ואם לא ישארו כראשונים דע בודאי שטעית ותחזור ותחשוב בטוב | ||||||||||||||||||||||||||
|
מאזנים אחרים על דרך השבעה | ||||||||||||||||||||||||||
|
תחשוב כל המדרגות כאשר הם ר"ל האלפים אלפים והמאות מאות והעשרות עשרות והאחדים אחדים לא שתחשבם כאלו הם אחדים כאשר עשית בדרך התשעה | ||||||||||||||||||||||||||
|
ואחר שתחשבם השליכם ז' ז' והשאר שמרהו | ||||||||||||||||||||||||||
|
ואם לא ישאר דבר שמור שמור בלבך סיפרא | ||||||||||||||||||||||||||
|
אחר תבא אל מדרגות הסך ותתחיל מהכלל היותר גדול עם מה שבצדו ותחשוב כאלו הכלל הם עשרות ומה שבצדו אחדים והשליכם ז' ז' ומה שישאר תחשבהו עשרות וחברהו עם מה שבצדו ותחשוב מה שבצדו לאחדים והשליכם ז' ז' וכן תעשה עד שתגיע אל האחדים וכשתגיע אליהם והשלכתם ז' ז' ראה הנותר ואם הוא שוה עם מה ששמרת אפשר שחשבונך יהיה אמתי ואם הוא בלתי שוה דע בודאי שטעית ותחזור ותחשוב בטוב | ||||||||||||||||||||||||||
|
וכן אם חלקת הטורים לחלקים מצד רבויים כאשר הזכרתי ועשית הסך חלקים חלקים תעשה גם המאזנים [שלהם][17] ככה אחר חבר גם המאזנים ככה כמו שתחבר הסכום על הדרך אשר ידעת |
Chapter Three: Subtraction |
הפרק השלישי | |||||||||||||
| If you want to subtract a number from a number, the whole number from which you subtract must be greater than the number you subtract, this with regard to integers, but not to fractions, and for the other ranks it is not necessary [except for the highest rank]. | אם רצית לחסר מספר ממספר ראוי שיהיה כלל המספר אשר תחסר ממנו יותר גדול מהמספר אשר תחסר וזה בשלמים אך לא בשברים ובשאר המדרגות אין צורך | |||||||||||||
| Always write the number from which you subtract first, then write the number you subtract beneath it, each rank beneath its corresponding rank. | ולעולם תכתוב המספר אשר תחסר ממנו ראשונה אחר תכתוב תחתיו המספר אשר תחסר כל מדרגה תחת הדומה לה | |||||||||||||
| If a rank is missing, write a zero in its place and always be careful not to forget the zero, but put it wherever a rank is missing. | ואם יש לשם חסרון מדרגה תכתוב סיפרא במקומה [18]והזהר לעולם אל הסיפרא שלא תשכחנה אך בכל מקום שתחסר מדרגה תשימנה | |||||||||||||
| Then, start subtracting from the units: | אחר כן תתחיל לחסר מהאחדים | |||||||||||||
|
ואם האחדים אשר תחסר מהם הם יותר מאלה אשר תחסר חסרם מהם והנשאר תכתוב למטה במדרגת האחדים | |||||||||||||
|
ואם הם פחות מהם תחזור אל העשרות אשר בצדם וקח עשירית אחת וחסרה מן העשרות וחברה עם האחדים אחר גרע מהם מה שתגרע והשאר תכתבם למטה במדרגת האחדים | |||||||||||||
| Then subtract the tens from the tens: | עוד תבוא לחסר העשרות מהעשרות | |||||||||||||
|
ואם יותר העשרות שתחסר מהם חסר מהם והשאר כתבם תחת העשרות | |||||||||||||
|
ואם הם מעטים שוב אל המאות | |||||||||||||
| Proceed according to the rule, until you reach the highest rank. | ועשה כמשפט עד שתגיע אל הכלל היותר גדול | |||||||||||||
| If there is a zero there and you want to subtract from it what is beneath it, take [one] from the rank that is higher than it. | אמנם אם יש לשם סיפרא ותרצה לחסר ממנה מה שלמטה ממנה תקח מהכלל אשר למעלה ממנה | |||||||||||||
| This is the diagram: | וזאת היא הצורה | |||||||||||||
|
נרצה לחסר ד' אלפים ומאתים ועשרים וחמשה מה' אלפים ומאה וארבעה עשר | |||||||||||||
| ||||||||||||||
|
הוצאנו החמשה מן הארבעה ומפני שהארבעה פחותים מהם חזרנו אל העשרות אשר למעלה מהם ומצאנו עשירית אחת ולקחנוה ועשינוה עשרה וחברנוה עם הארבעה ונהיו ארבעה עשר הוצאנו מהם החמשה ונשארו תשעה וכתבנום במדרגת האחדים | |||||||||||||
|
עוד רצינו לגרוע העשרים מן הסיפרא כי לא נשאר דבר מהעשרות וחזרנו אל המאות והיה מאה אחת ולקחנוה והחזרנוה אל עשר עשרות והוצאנו ממנה העשרים ונשארו שמנים וכתבנום למטה | |||||||||||||
|
עוד רצינו לגרוע המאתים מהסיפרא כי לא נשאר לנו במאות דבר וחזרנו אל האלפים ולקחנו אלף אחת מן האלפים ונשארו האלפים ד' והאלף אשר לקחנו החזרנוה עשר מאות וגרענו מהם המאתים ונשארו שמנה מאות וכתבנום במדרגת המאות | |||||||||||||
|
עוד גרענו הד' אלפים מד' אלפים ונשאר 0' | |||||||||||||
|
אם כן ידענו שכשחסרנו ד' אלפים ורכ"ה מה' אלפים וקי"ד נשארו סך סיפרא אלפים ושמנה מאות ושמנים ותשעה | |||||||||||||
Check |
||||||||||||||
|
מאזנים על דרך התשעה | |||||||||||||
|
תחשוב כל המדרגות שגרעת מהם כאלו הם אחדים ותשליכם ט' ט' ומה שישאר שמרהו ויקרא השמור הראשון | |||||||||||||
|
וכן תעשה במספרים אשר גרעת אותם שתשמור מהם הנשאר מהט' ט' וזה יקרא השמור השני | |||||||||||||
|
אחר תגרע השמור השני מהראשון ומה שישאר שמרהו וזה יקרא השמור השלישי | |||||||||||||
|
עוד תעיין אל הסך הנשאר אחר שגרעת המספרים מהמספרים ותחשבם כאלו הם אחדים והשליכם ט' ט' והנשאר אם יהיה שוה לשמור השלישי אפשר שחשבונך יהיה אמת ואם אינו שוה דע בודאי שטעית | |||||||||||||
|
ואם השמור הראשון פחות מהשמור [השני][19] תוסיף עליו ט' אחר תגרע השמור השני ממנו ותעשה כמשפט | |||||||||||||
|
מאזנים אחרים על דרך השבעה | |||||||||||||
|
תחשוב כללי המספרים אשר גרעת מהם עשרות [20]ואשר בצדם אחדים והשליכם ז' ז' ומה שישאר תחשבהו עשרות והמספר הבא אחריו אחדים והשליכם ז' ז' עד שתגיע אל האחדים והשליכם ז' ז' ומה שישאר שמרהו וזה יקרא השמור הראשון | |||||||||||||
|
עוד תעשה ככה במספרים אשר גרעת אותם והנשאר שמרהו ויקרא השמור השני | |||||||||||||
|
גרע השמור השני מהשמור הראשון ואשר [ישאר][21] יקרא השמור השלישי | |||||||||||||
|
אח"כ תעיין אל המספרים אשר נשארו כשגרעת המספרים מהמספרים וחשבם ככה כאשר אמרנו בטורים הראשונים כלומר כשתחשוב הכללים עשרות ואשר בצדם אחדים והשליכם ז' ז' והנשאר תחשבהו עשרות ואשר בצדו לאחדים ותשליכם ז' ז' עד שתגיע אל האחדים ואחר שהשלכתם ז' ז' עיין בנשארים ואם הם שוים לשמור השלישי אפשר שחשבונך יהיה אמת ואם לא דע כי בודאי טעית | |||||||||||||
|
מאזנים אחרים על דרך הקבוץ והם מאזני צדק | |||||||||||||
|
חבר המספרים שגרעת אותם עם המספרים שנשארו אחר הגרעון וראה ואם יהיו שוים עם המספרים אשר גרעת מהם תדע בודאי כי חשבונך אמת ואם לא בודאי טעית | |||||||||||||
| The first part is complete. | [תם החלק הראשון][22] |
Part Two: Multiplication |
החלק השני מהספר הראשון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
The First Chapter: To Multiply a Number by a Number |
הפרק הראשון לכפול מספר על מספר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Whoever wants to multiply a number by a number should memorize this table, because by that he can multiply quickly a certain number by a certain number from 1 to 9. | הרוצה לכפול מספר על מספר ראוי שיהיה הלוח הזה שגור בפיו כי בזה יהיה זריז לכפול מספר פלוני על מספר פלוני מא' עד ט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If the numbers he wants to multiply by [each other] are each of one rank, or whichever rank they are, he takes for each their analogous in the rank of units, multiply one by the other as if they are units and see the result. | ואם יהיה המספר אשר ירצה לכפול על מספר אחר כל אחד ממדרגה אחת או מאיזו מדרגה שיורה יקח דמיון לכל אחד ממדרגת האחדים ויכפול זה על זה כאלו הם אחדים ויראה העולה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Then, he takes for each the value of its rank: if the one is of the tens and the other is of the hundreds, we take two for the tens and three for the hundreds. We sum them; it is five. | ואח"כ יקח לכל האחד מספר במדרגתו שאם היה הא' עשרות והאחר מאות נקח בעד העשרות שנים ובעד המאות שלשה ונחברם ויהיו חמשה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| We always subtract one and the remainder indicates for us of what rank [the product] is. | אח"כ נשליך לעולם אחד והשאר יורה לנו העולה מאיזה מדרגה הוא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון בזה רצינו לכפול שמנים על חמש מאות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
לקחנו דמיונם מהאחדים בעד השמני' שמנה ובעד החמש מאות חמשה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכפלנו חמשה על שמנה ויצא לנו הסכום ארבעים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד לקחנו בעד המאות שלשה ובעד השמנים שנים כי הם מהמדרגה השנית חברנום ונהיו חמשה השלכנו אחד ונשארו ארבעה ואלה הארבעה הם מדרגת האלפים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וידענו מזה שהארבעים אשר יצאו כשכפלנו השמנים על החמש מאות הם ארבעים אלף | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Yet, if you want to multiply two digits by two digits, we should write each of them in its rank and multiply them four times, each one of the bottom line by each one of the upper line. | אולם כשתרצה לכפול שני מספרים על שני מספרים צריך שנכתוב אותן כל אחד במדרגתו ושנכפול אותן ארבעה פעמים כל אחד מהטור שלמטה עם כל אחד מהטור שלמעלה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| We write the given number in the first line and the number, by which we want to multiply it, in the bottom line. | ונכתוב המספר המונח בטור הראשון והמספר אשר נרצה לכפול עליו בטור התחתון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| We start multiplying the units of the bottom [line] by the units of the upper [line]. | ונתחיל לכפול אחדי התחתון על אחדי [23]העליון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| We write the result beneath in the rank of units, if it does not reach tens. | וההוה נכתוב אותו למטה במדרגת האחדים וזה אם לא הגיע לעשרות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| But, if it reaches tens, we take out the tens and keep them. We write the remaining that does not reach ten in the rank of units. | אבל אם הגיע לעשרות נוציא העשרות מהם ונשמרם והנשארים אשר לא הגיעו לכלל העשרה נכתוב במדרגת האחדים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| We also multiply the units below by the rank of tens above. | עוד נכפול האחדים שלמטה עם מדרגת העשרו' שלמעלה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| We add the number of tens we kept to the units of this product, because although they are tens, the result is as if they are tens and units, for we multiply everything as units. | ואשר יהיה נחבר גם מספר העשרות ששמרנו עם אחדי זה הכפל כי אע"פ שהם עשרו' אבל מה שיצא מהם יהיה כאלו הם עשרות ואחדים כי על דמיון האחדים אנו כופלים הכל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| We write the units resulting from this multiplication in the rank of tens and we write the tens in the third rank. | והאחדים אשר יתקבצו מזה הכפל נכתבם במדרגת העשרות והעשרות אם יהיו נכתבם במדרגה השלישית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Then, we look at the result and this is the product of this number. | אחר נראה העולה והוא חשבון כפל המספר ההוא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון רצינו לכפול עשרים וחמשה על ארבעים ושלשה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| כזה | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפלנו על הג' הה' ונהיו ט"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
גרענו העשירית ושמרנוה נשארו ה' וכתבנו אותם במדרגת האחדים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד כפלנו הה' על הד' ונהיו עשרים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומפני שלא יצאו מזה אחדים כתבנו העשירית האחת ששמרנו במקום העשרות והעשרים כתבנום במדרגה השלישית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד כפלנו הב' על הג' ונהיו ששה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומפני שלא יצאו גם בכאן אחדים כתבנו במקום [האחדים][24] סיפרא והששה כתבנום במקום העשרות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד כפלנו העשרים על הארבעי' ונהיו שמנה מאות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכתבנום במדרגה השלישית כי כל אחד מהכפולים היו ממדרגת שנית ובהשלכת האחד נשארו שלשה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אחר כן קבצנום ונהיו אלף ושבעים וחמשה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If we want to multiply three digits by three digits, we should multiply them nine times by this order. | ואם נרצה לכפול שלשה מספרים על שלשה מספרים אז ראוי שנכפלם תשעה פעמים בזה הסדר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון רצינו לכפול שלש מאות וארבעים ושמנה על מאתים ושלשים וחמשה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כתבנו אותם בצורה הזאת | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפלנו הח' על הה' ונהיו ארבעים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומפני שאין כאן אחדים כתבנו סיפרא במדרגת האחדים ושמרנו העשרות הארבעה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד כפלנו הג' עם הח' ונהיו כ"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שמרנו שתי עשרות ועם הארבעה שהם אחדיו חברנו הארבעה אשר שמרנו ונהיו שמנה וכתבנום במדרגת העשרות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד כפלנו הב' על הח' ונהיו י"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שמרנו העשירית האחת וחברנו עם אחדיו שהם הששה את שתי עשרות[25] שהיו שמורות לנו ונהיו ח' וכתבנום במדרגת המאות והעשירית האחת אשר שמרנו עתה כתבנוה במדרגת האלפים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה כבר כפלנו הח' על כל אחד ואחד מהמספרים שבטור הראשון השלשה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד שבנו לכפול הד' עם כל אחד ואחד מהם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונכפול תחלה עם הה' ונהיו עשרים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומפני שלא יצאו אחדים כתבנו ספרא במדרגת העשרות שהיא מדרגת אחדיו כי המספרים שכפלנו הם ממדרגה הראשונה והשנית והעשרים שהם שני עשרות שמרנום | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד כפלנו הד' עם הג' ונהיו י"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שמרנו העשירית האחת וחברנו [26]עם השנים שהם אחדיו השני עשרות שהיו שמורות לנו ונהיו ד' כתבנום במדרגת המאות לפי שהמספרים שכפלנו הם במדרגה השניה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד כפלנו הארבעה עם הב' ונהיו ח' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חברנו גם העשירית השמורה לנו עמם ונהיו ט' כתבנום במדרגת האלפים לפי שהמספרי' אשר כפלנו היו ממדרגה השנית והשלישית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה כבר כפלנו גם הד' שבטור השפל עם כל אחד ואחד מהשלש מספרים שבטור העליון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד נשוב לכפול הג' עם כל אחד ואחד [מן][27] שלשת המספרים העליונים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפלנו אותו עם ה' והיה ט"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הוצאנו העשירית ושמרנוה והה' כתבנום למטה במדרגת המאות כי שני המספרים האלה מדרגותם הם הראשונה והשלישית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד כפלנו אותם עם ג' ונהיו ט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חברנו גם העשירית השמורה ונהיו י' ולא היו לשם אחדים ולכן כתבנו במדרגת האלפים ספרא והעשירית אנו שומרים אותה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד הכינו אותם עם שנים ונהיו ו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חברנו גם העשירית השמורה עמם ונהיו ז' וכתבנום במדרגת הרבבות כי שני המספרים האלה במדרגה השלישית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אחרי כן קבצנו שלשה הסכים כמשפט ונהיו פ"א אלף ושבע מאות ושמנים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון אחר במספר שיש לו ספרא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
רצינו לכפול מאה וחמשה על מאתים ועשרים וארבעה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כתבנו אותם ככה בזאת הצורה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפלנו הה' על הד' ונהיו כ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומפני שאין שם אחדים כתבנו סיפרא במדרגת האחדים והשתי עשרות שמרנום | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד כפלנו הה' עם הב' ונהיו י' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומפני שאין שם אחדים כתבנו [הב' עשרות השמורות במדרגת העשרות וזאת העשירית שמרנוה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד כפלנו הה' עם הב' ונהיו י' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומפני שאין שם אחדים כתבנו][28] למטה העשירית אשר שמרנו במדרגת המאות וזאת העשירית במדרגת האלפים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד שבנו להכות כל אחת משלשה המספרים העליונים עם הסיפרא וכתבנו סיפרא במדרגת העשרות וכן במדרגת המאות וכן במדרגת האלפים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד שבנו לכפול הא' עם הד' ונהיה ד' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כתבנו אותו במדרגת המאות כי שני המספרים הנכפלים היו מהמדרגה הראשונה והשלישי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד כפלנו הא' עם הב' ונהיו ב' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכתבנום במדרגת האלפים כי שני המספרי' הכפולי' היה האחד מהמדרגה השנית והאחר מהמדרגה השלישית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד כפלנו הא' עם השנים ונהיו ב' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכתבנום במדרגת הרבבות כי שני המספרים הכפולים היה כל א' מהמדרגה השלישית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אחר כן קבצנו העולה מהם והם כ"ג אלף וחמש מאות ועשרים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Said Mordecai: I saw fit to teach you another easier way I have found among the Arab sages, in which you write the whole product in one line without the need for more than that. | אמר מרדכי ראיתי להודיעך הכפל הזה בדרך אחרת נקלה מצאתיה אצל חכמי הישמעאלים והוא שתעשה כל סך הנכפלים בשטה אחת מבלתי שתצטרך אל יותר מזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Do as follows: | וככה תעשה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Write the number you want to multiply in the upper line as usual. | תשים המספר אשר תרצה לכפול עליו בשטה העליונה כמנהג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Write also the number by which you want to multiply it in the bottom line, each rank corresponding to its similar [rank]. | עוד שים המספר אשר תרצה לכפול אותו עליו בשטה השפלה כל מדרגה כנגד הדומה לה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Then, start to multiply the units by the units and write the result below. | ואחר כן החל לכפול האחדים על האחדים ותכתוב [29]העולה למטה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If tens are received, keep them and write only the units below. | [ואם יעלו עשרות תפוש אותם בלבך ותכתוב האחדים לבדם למטה][30] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Multiply also the units that are in the bottom line by the tens that are in the upper line as if they are units by units. | עוד תכפול האחדים שבשטה השפלה עם העשרות שבשטה העליונה כאלו הם אחדים על אחדים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If you are left with tens from the first product, consider them as units and add them to these. | ואם נשארו לך עשרות מהחשבון הראשון חשוב אותם אחדים וחברם עם אלה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Multiply also the units that are in the upper line by the tens that are in the second line. | גם תכפול האחדים שהם בשטה העליונה עם העשרות שהם בשטה השניה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Sum up all and write the result . | וחבר הכל ותכתוב העולה למטה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If tens are received, meaning of a higher rank than the present rank, keep them. | ואם עלו עשרות והטעם מדרגה עליונה מזאת תפוש בלבך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Multiply also the units that are in the bottom line by the hundreds that are in the upper line. | גם תכפול האחדים שבשטה השפלה עם המאות שבשטה העליונה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Also the units that are in the upper line by the hundreds that are in the bottom line. | [וכן האחדים שבשטה העליונה][31] עם המאות שבשטה השפלה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Also the tens that are in the bottom line by the tens that are in the upper line. | גם העשרות שבשטה השפלה עם העשרות שבשטה העליונה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The rule: the sum of [the positional value of the] ranks is equal to [the positional value of] the product. | והכלל המדרגות אשר בקבוצן תהיינה שוות הסך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כי הראשונה עם השלשה יעשו ד' וככה השלישית עם הראשונה והשנית עם השנית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
גם הראשונה עם הרביעית יעשו חמשה וככה השנית עם השלישית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Proceed constantly and add to it the number you kept that is in the rank we consider as tens. | וככה תעשה עד אין קץ ואשר יהיה תחבר המספר שהיה במדרגה שחשבנוה עשרות שתפשת בלבך עמם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Write them beneath and it is the required number. | וכתבם למטה והוא הסך המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון רצינו לכפול שלש מאות וארבעים ושמנה על מאתים ושלשים וחמשה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| כזה | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפלנו הח' על הה' ונהיו מ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ובעבור שלא נשארו אחדים כתבנו גלגל במדרגה הראשונה והמ' תפשנום בלבנו ארבע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד כפלנו ח' עם ג' והיו כ"ד גם ד' עם ה' כי הם שוה המדרגות והיו כ' חברנום עם הכ"ד והיו מ"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
גם חברנו עמהם הד' שתפשנו בלבנו היו מ"ח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכתבנו למטה במדרגת העשרות ח' והמ' תפשנו בלבנו ד' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד כפלנו ח' עם ב' והיו י"ו גם ג' עם ה' והיו ט"ו גם ד' עם ג' והיו י"ב כי כל אלה שוה המדרגות והם מ"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חברנו עמהם הד' שתפשנו בלבנו והם מ"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כתבנו הז' במדרגת המאות והד' שהם המ' תפשנו בלבנו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד כפלנו ד' עם ב' והיו ח' גם כפלנו ג' עם ג' והיו ט' גם חברנו הד' שתפשנו בלבנו עמם והיו כ"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כתבנו הא' במדרגת האלפים והכ' תפשנו אותם בלבנו שנים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד כפלנו ג' עם ב' והיו ו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חברנו עמהם הב' שתפשנו בלבנו והיו ח' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כתבנו הח' במדרגת הרבבות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וידענו מזה שהסך העולה מכפל השטה השפלה על השטה העליונה הם שמנים ואחת אלפים ושבע מאות ושמנים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Shortcuts |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The arithmeticians tend to multiply by a third method: | וכבר נהגו בעלי החשבון לכפול בדרך שלישית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| It is that you take the third of the number, multiply it by itself, the product is the square [of its third], take its analogous in the next rank that is higher than [the present rank], then subtract the square of the third from this rank and the remainder is the required.
|
והוא שתקח שלישית המספר ותכפלהו על עצמו וההוה הוא מרובעו ותקח כדומה לו ממדרגה הבאה אשר היא יותר עליונה ממנה ותוציא מזאת המדרגה מרובע השלישית והנשאר הוא המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון רצינו לדעת מרובע ט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולקחנו שלישיתו וכפלנו אותו על עצמו ונהיה ט' לקחנו דמיונו ממדרגה הבאה העליונה ממנה והיה צ' הוצאנו ממנו הט' שהוא מרובע השלישית ונשארו פ"א והוא המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If you want to multiply a number that does not have a third, you take a third of the closest number to it, square it and do according to the rule, then examine if the number, whose third you took, is less than the required number, sum up the two numbers and add them to the amount; the result is the wanted.
|
ואם רצית לכפול מספר שאין לו שלישית אתה לוקח שלישית המספר יותר קרוב אליו ותרבע אותו ותעשה כמשפט אחר תעיין ואם המספר אשר לקחת שלישיתו הוא יותר פחות מהמספר המבוקש קבץ שני המספרים והוסיפם על הסך וההוה הוא המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון רצינו לדעת מרובע עשרה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
[32]וזה אין לו שלישית והמספר הקרוב אליו אשר יש לו השלישית הוא התשעה והוא פחות ממנו לקחנו מרובע שלישיתם והוא ט' ולקחנו דמיון מהמדרגה הבאה והוא צ' גרענו ממנו ט' ונשארו פ"א ומפני שהמספר אשר לקחנו השלישית ממנו הוא פחות מהמספר המבוקש קבצנו שני המספרים הט' עם הי' ונהיו י"ט הוספנום על הפ"א ונהיו ק' והוא מרובע העשרה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| But, if the number, whose third we took, is greater than the required number, we sum up the two numbers and subtract them from the amount; the remainder is the wanted.
|
אבל אם היה המספר אשר לקחנו השלישית ממנו יותר מהמספר המבוקש אנו מקבצים שני המספרים וגורעים אותם מהסך והנשאר הוא המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון רצינו לדעת מרובע י"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומפני שאין לו שלישית והמספר הקרוב אליו שיש לו שלישית הוא הי"ב והוא יותר ממנו לקחנו שלישיתו וכפלנוהו במספר המרובע ולקחנו דמיונו במדרגה הבאה והוא ק"ס גרענו ממנו הי"ו שהוא מרובע השלישית ונשארו קמ"ד ומפני שהמספר אשר לקחנו השלישית ממנו שהוא י"ב הוא יותר מהמספר המבוקש מרבעו שהוא י"א קבצנו שתיהן ונהיו כ"ג וגרענו אותם מן קמ"ד ונשארו קכ"א וזהו המבוקש שהוא מרובע י"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Said Mordecai: I have found a respectable way of multiplication by the method of fifths: | [אמר מרדכי][33] מצאתי דרך נכבד בענין הכפל בדרך החמישיות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| That is when you wish to multiply a number by itself, take its fifth, multiply it by itself, raise it to the next rank, then multiply it by two and a half and this is the required.
|
והוא כי כאשר תרצה לכפול מספר על עצמו תקח חמישיתו ותכפלהו על עצמו ותעלהו במעלה הבא הסמוכה לה אחר תכפול אותה על שנים וחצי והוא המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון רצינו לכפול ששים על ששים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וחמישיתו י"ב כפלנום על עצמם נהיו קמ"ד העלנו אותם במדרגה הבאה הסמוכה להם נהיו אלף וארבע מאות וארבעים כפלנום על ב' וחצי ונהיו ג' אלפים ת"ר וזהו המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון רצינו לכפול חמשים על חמשים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וחמישיתו עשרה כפלנוהו על עצמו ונהיה מאה העלינוהו אל המדרגה הסמוכה לה ונהיו אלף כפלנום על ב' וחצי ונהיו ב' אלפים וחצי וזהו המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If you want to multiply a number by itself, but it does not have a fifth. Examine the closest number to it that has a fifth, whether preceding it or following it. The difference never exceeds over two. | ואם רצית לכפול מספר על עצמו ואין לו חמישית תעיין המספר הקרוב אליו שיש לו חמישית אם מלפניו או מלאחריו ולא יעבור ההפרש לעולם על שנים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If the closest number to your number that has a fifth precedes your number by one, calculate it by the method I have mentioned first regarding the number that has a fifth, then sum up your number with the number that has a fifth, add it to the amount and this is the required.
|
ואם היה המספר שיש לו חמישית הקרוב אל מספרך [קודם ממספרך][34] באחד תחשבהו בדרך שהזכרתי ראשונה על המספר שיש לו חמישית אחר תחבר מספרך עם המספר שיש לו חמישית ותוסיפהו על הסך והוא המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If the difference is two, multiply your number, after you sum it up with the number that has a fifth, by two, then add it to the amount and this is the required.
|
ואם היה ההפרש שנים תכפול מספרך אחר שתחברהו עם המספר שיש לו חמישית עם שנים ואחר תוסיפנו על הסך והוא המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If the number that has a fifth follows your number, calculate your it using the number that has a fifth, then add your number to the number once, if the difference is one, or multiply it by 2 after you sum [them] up, if the difference is two. Subtract [the sum] from the amount and the remainder is the required. | אמנם אם המספר שיש לו חמישית הוא אחר מספרך תחשוב חשבונך על המספר שיש לו החמישית אחר תחבר מספרך עם המספר פעם אחת אם ההפרש אחד או תכפול אותו עם ב' אחר שחברתו אם ההפרש שנים ותגרעהו מהסך והנשאר הוא המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון רצינו לכפול י"א על י"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה זה המספר אין לו חמישית והמספר הקרוב אליו אשר יש לו חמישית הוא העשרה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכפלנו אותו בדרך החמישיות ועלו ק' אחר חברנו הי' שיש לו החמישית עם הי"א [35]שהוא מספרנו פעם אחת מפני שההפרש בין מספרנו ובין י' הוא אחד ונהיו כ"א חברנו עם הק' ונהיו קכ"א וזהו המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Another example of a number, such that the difference is two: | דמיון אחר במספר שההפרש שנים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
רצינו לכפול י"ב על י"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה זה המספר אין לו חמישית והמספר הקרוב לו בעל החמישית הוא העשירי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפלנוהו בדרך החמישיות ונהיו ק' אחר חברנו הי' שיש לו החמישית עם הי"ב שהוא מספרנו ונהיו כ"ב ומפני שההפרש שנים כפלנום ב' פעמים ונהיו מ"ד וחברנום עם הק' והיו קמ"ד וזהו המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Other examples of numbers, such that the closest number to them that has a fifth comes after them, because in the examples we gave it precedes them: | דמיון אחר במספרים אשר המספר שיש לו חמישי' הקרוב אליו הוא אחריהם כי הדמיונים אשר עשינו היה בהיותו לפניהם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
רצינו לכפול י"ד על י"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה זה המספר אין לו חמישית ראינו המספר אשר יש לו חמישית הקרוב אליו והוא ט"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפלנום בדרך החמישיות ועלה רכ"ה אחר חברנו את המספר הזה שיש לו חמישית עם מספרנו שהוא י"ד פעם אחת מפני שההפרש אחד והיה כ"ט גרענום מן הסך מפני כי המספר אשר יש לו החמישית היה אחרי מספרנו ונשארו קצ"ו וזהו המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Example of a number, such that the difference between it and the [number] that has a fifth is two: | דמיון במספר כזה שההפרש בינו ובין אשר יש לו החמישית שנים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
רצינו לכפול י"ג על י"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה המספר אין לו חמישית והמספר הקרוב שיש לו החמישית הוא החמשה עשר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפלנוהו בדרך החמישיות והיה רכ"ה אחר חברנו זה המספר שיש לו החמישית עם י"ג שהוא מספרנו ונהיו כ"ח כפלנום על ב' מפני שההפרש בין שני המספרים ב' ונהיו נ"ו גרענום מן רכ"ה מפני שהמספר שיש לו החמישית הוא אחרי מספרנו ונשארו קס"ט וזהו המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If you want to multiply a number by a number that are far from the kelal by the same amount, one smaller than it and the other exceeding it, you can multiply the kelal by itself, then square the excess and subtract it from the product of the kelal; the result is the required. | ואם תרצה לכפול מספר על מספר שהם רחוקים מהכלל בשווי האחד חסר ממנו והאחר מוסיף עליו אתה יכול לכפול הכלל על עצמו [אחר][36] תרבע המספר היתר והחסר ותגרעהו מחשבון הכלל וההוה הוא המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון רצינו לכפול כ"ה על ל"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והכלל ל' והה' בתוספת וחסרון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפלנו הכלל ונהיו תת"ק אחר כפלנו הה' ונהיה כ"ה גרענום מהתת"ק ונשארו תתע"ה והוא המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Said Mordecai: although we have said that if the numbers are two [digits] by two [digits], you should multiply them four by four, i.e. four times [= four interim products], you should know that if their kelal is the same, it is enough to multiply them three times [= three interim products]. | אמר מרדכי עם היות שאמרנו שאם היו המספרים שתים על שתים אתה צריך לכפלם ארבעה על ארבעה ר"ל ארבעה פעמים צריך אתה לדעת שאם היה כללם אחד יספיק לכפלם שלש פעמים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כגון הרוצה לכפול י"ג על י"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה כלל שניהם עשרה תחבר שני הפרטים ויהיו ז' ותכפול ז' פעמים י' נהיו ע' וי' פעמים י' הרי ק' וג' פעמים ד' הרי י"ב והעולה קפ"ב וזהו המבוקש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Check |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Scales of multiplication by casting out nines [lit. by the Nine way]: | מאזנים על הכפל בדרך הט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תחשוב הטור העליון כולו כאלו הם אחדים ותשליך אותם ט' ט' והשאר שמרהו וזה יקרא השמור הראשון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד תעשה כן בטור השפל והשאר יקרא השמור השני | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תכפול השמור הראשון על השמור השני [ואשר יהיה השליכהו ט' ט'][37] והשאר שמרהו וזה יקרא השמור השלישי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אחר תעיין הטור השלישי השפל אשר יקרא הסך ותחשבהו כאלו הם אחדים והשליכם ט' ט' [38]והשאר עיין ואם הוא שוה לשמור השלישי אפשר שחשבונך יהיה אמת ואם לאו דע בודאי שטעית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Scales by casting out sevens [lit. by the Seven way]: | מאזנים בדרך השבעה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עיין מספרי הטור הראשון ותתחיל מהיותר כולל ותחשבהו עשרות ואשר בצדו אחדים והשליכם ז' ז' ואשר ישאר עוד תחשבהו עשרות ואשר בצדו אחדים והשליכם ז' ז' וכן תעשה [עד שתגיע][39] למעלת האחדים ואשר ישאר שמרהו וזה יקרא השמור הראשון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד תקח מספרי הטור השני ועשה כמשפט הזה ואשר ישאר יקרא השמור השני | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אחר תכפול השמור השני על השמור הראשון ואשר יהיה השליכהו ז' ז' והשאר יקרא השמור השלישי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אחר כן תעיין מספרי הטור השפל השלישי אשר יקרא הסך ועשהו כמשפטי הטורים העליונים ועיין הנשאר ואם הוא שוה לשמור השלישי אפשר שחשבונך יהיה אמת ואם לאו דע בודאי שטעית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Euclidean Propositions |
|
| Said Mordecai: I thought to write you some issues of number to train you to be be quick in these matters. | אמר מרדכי ראיתי לכתוב לך דברים מעניני המספר להרגילך בהם כדי שתהיה זרִיז בענינים אלו |
| Euclid, Elements, Book II, propositions 2: I say: if you divide any number into parts as you wish, [the sum of] the products of each of the parts by the whole number is equal to the square of the whole number.
|
ואומר כל מספר שחלקת אותו לחלקים איך שרצית הנה כפל כל אחד מהחלקים על כל המספר מקובץ שוה למרובע כל המספר |
|
דמיון המספר י"ב וחלקנוהו על שלשה וארבעה וחמשה |
|
כפלנו הג' על הי"ב והיו ל"ו |
|
עוד כפלנו הה' על הי"ב והיו ס' |
|
עוד כפלנו הארבעה על הי"ב והיו מ"ח |
|
חברנו אותם והיו הכל קמ"ד והם שוים למרובע י"ב |
| Euclid, Elements, Book II, proposition 2: For every number that you divide randomly into two parts, the sum of the products of each of the two parts by the whole number is equal to the square of the whole number.
|
עוד כל מספר שחלקת אותו לשני חלקים איך שקרה הנה כפל כל אחד משני החלקים על כל המספר מקובץ שוה למרובע כל המספר |
|
דמיון המספר עשרה וחלקנוהו על ז' וג' |
|
כפלנו הז' על הי' והיו שבעים |
|
גם ככה כפלנו החלק האחר שהם השלשה עליו והיו ל' |
|
ושניהם ק' והוא מרובע העשרה |
|
עוד כל מספר שחלקת אותו בשני חלקים איך שקרה הנה כפל המספר כולו על אחד משני חלקיו איזה שיהיה שוה לכפול החלק האחד על השני ולמרובע החלק משניהם אשר כפלת על כל המספר |
|
דמיון המספר עשרה חלקנוהו לשני חלקים על שלשה ושבעה |
|
כפלנו השלשה עמו והיו ל' וזה שוה לכפל הג' על השבעה שהם כ"א ולמרובע הג' שהם ט' שהוא החלק אשר כפלנו |
|
גם ככה אם היינו כופלים הז' שהוא החלק [האחד][40] על העשרה היו שבעים וזה שוה לכפל הג' על הז' שהם כ"א ולמרובע הז' שהם מ"ט שהוא החלק אשר כפלנו ושניהם שבעים |
|
עוד כל מספר שחלקת אותו לשני חלקים איך שקרה הנה מרובע כל המספר שוה לשני המרובעים ההוים משני החלקים ולכפל החלק האחד על חברו פעמים |
|
דמיון המספר עשרה חלקנוה על שלשה ושבעה |
|
ומרובע הג' ט' ומרובע הז' מ"ט וכפל הג' על הז' כ"א וחשבהו פעמים הם מ"ב והכל ק' וזה שוה למרובע העשרה |
|
| |
|
עוד כל מספר כאשר תחלקהו לשני חלקים שוים ולשני חלקים בלתי שוים הנה כפל החלק האחד אל חברו מהחלקים הבלתי שוים ומרובע מה שבין שני [41]החלקים ר"ל בין החלק השוה ובלתי שוה שוה למרובע חצי המספר |
|
דמיון המספר עשרה חלקנו לחמשה וחמשה שהם חלקים שוים גם חלקנוהו לז' וג' שהם חלקים בלתי שוים |
|
כפלנו הג' על הז' והיו כ"א |
|
עוד לקחנו מרובע השנים שהם בין הה' שהם החלק השוה לשלשה או לז' שהם החלק הבלתי שוה והיו ד' |
|
והכל כ"ה והם שוים למרובע ה' שהוא מרובע חצי המספר |
|
| |
|
עוד כל מספר כאשר חלקת אותו לחצאים והוספת עליו מספר אחר הנה כפל המספר כלו מקובץ עם התוספת בתוספת והמרובע ההוה מחצי המספר שוה למרובע חצי המספר והתוספת ביחד |
|
דמיון המספר עשרה וחלקנוהו לשני חצאים שהם כל חצי חמשה הוספנו על העשרה שנים והיו י"ב |
|
כפלנו כל הי"ב שהוא המספר עם התוספת ביחד עם השנים שהם התוספת והיו כ"ד |
|
חברנו עמהם כ"ה שהם מרובע ה' [שהוא][42] חצי המספר והיו מ"ט וזה שוה למרובע ז' שהוא חצי המספר עם התוספת ביחד |
|
| |
|
עוד כל מספר כשתחלקהו בשני חלקים איך שיקרה הנה המרובע ההוה מן המספר כלו והמרובע ההוה מאחד משני חלקים כאשר התקבצו שוים לכפל המספר כלו עם החלק הנזכר פעמים והמרובע ההוה מן החלק השני |
|
דמיון המספר עשרה וחלקנוהו איך שקרה על שבעה ועל שלשה |
|
והמרובע ההוה מעשרה הם ק' וההוה מז' הם מ"ט ומקובצים קמ"ט והם שוים לכפל העשרה על ז' פעמים שהם מאה וארבעים ולמרובע ג' שהם ט' שהוא החלק השני והכל קמ"ט |
|
| |
|
עוד כל מספר כאשר חלקת אותו בשני חלקים איך שיקרה וכפלת המספר כלו עם אחד משני חלקיו ארבעה פעמים ועם מרובע החלק הנשאר שוה למרובע ההוה מן המספר כלו והחלק הנזכר כאשר תחברם ביחד ותקח מרובעם |
|
דמיון יש לנו מספר מנינו העשרה וחלקנוהו איך שהזדמן על ג' ועל ז' |
|
הנה כאשר כפלנו הז' עם הי' ד' פעמי' היו ר"פ וכאשר חברנו עמהם מרובע הג' שהוא ט' והוא החלק השני נהיו רפ"ט והם שוים למרובע י"ז שהוא המספר כלו יחד עם החלק אשר כפלנוהו עם המספר כולו והם גם אלה רפ"ט |
|
עוד כל מספר שחלקת אותו לשני חלקים שוים ושני חלקים בלתי שוים הנה שני המרובעים אשר יהיו מהחלקים הבלתי שוים הם כפל שני המרובעים אשר יהיו מחצי המספר ומהתוספת אשר לחלק הגדול על המחצית |
|
דמיון יש לנו מספר מנינו עשרה וחלקנוהו לשני חלקים שוים על ה' ושני חלקים בלתי שוים על ג' וז' |
|
הנה מרובע ג' שהוא ט' ומרובע ז' שהוא מ"ט שניהם נ"ח הם כפל מרובע ה' שהוא כ"ה [והוא מחצית המספר][43] וכפל מרובע ב' שהוא ד' ושניהם כ"ט שהוא התוספת שיש לז' שהוא החלק הגדול על [הה' שהוא][44] המחצית |
|
| |
|
עוד כל מספר שחלקת אותו לשני חציים והוספת עליו מספר אחר הנה מרובע המספר עם התוספת יחד ומרובע התוספת בעצמו הם כפל שני המרובעים שהם מרובע חצי המספר ומרובע חצי המספר עם התוספת יחד כאשר יחוברו |
|
דמיון יש לנו מספר מניינו עשרה וחלקנוהו לשני חצאים על ה' והוספנו עליו [45]מספר אחר ב' ונהיה י"ב |
|
הנה מרובע י"ב שהוא המספר עם התוספת יחד קמ"ד |
|
ומרובע ב' שהוא התוספת ד' |
|
ושניהם קמ"ח והוא כפל מרובע ה' שהוא כ"ה ומרובע ז' שהוא מ"ט ושניהם ע"ד אשר הוא מרובע חצי המספר והתוספת ביחד |
|
| |
| I have informed you of useful proper ways of numbers, for if different ways lead to one calculation, when an error results from one way it can be corrected in the other way. Practice them. | הנה הודעתיך דרכים נאותים במספרים מועילים כי כאשר יהיו דרכים מתחלפים מוציאים אל חשבון אחד כאשר יטעה מהדרך האחד יתיישר מהדרך האחר ותרגיל עצמך בהם |
Part Three: To Divide a Number by a Number and to Sum a Number with a Number |
החלק השלישי לחלק מספר על מספר ולהוסיף מספר על מספר | |||||||||||||||||||||||||||||||||||||||||||||||||||||
Chapter One: Division |
הפרק הראשון | |||||||||||||||||||||||||||||||||||||||||||||||||||||
| Said Mordecai: We have preceded this chapter to the chapter on proportions, since it precedes proportion by nature and simpler than it. | אמר מרדכי הקדמנו זה השער משער הערכים להיותו קודם בטבע [מן הערכי' ופשוט ממנו | |||||||||||||||||||||||||||||||||||||||||||||||||||||
| It precedes by nature, because we can find in proportions one term of two or three only by multiplication and division, as will be explained in the chapter, in which we will mention them. | אם קודם בטבע][46] כי לא נוכל למצוא בערכים הגבול האחד מהשנים או מהשלשה אם לא בדרך הכפל והחלוק כאשר יתבאר בשער אשר נזכירם | |||||||||||||||||||||||||||||||||||||||||||||||||||||
| It is simpler than it, since that chapter consists of this [chapter] and of what is distinctive for it, while this chapter is more specific to both. | ואם פשוט ממנו להיות השער ההוא מורכב מזה ומהמיוחד לו וזה השער מיוחד בזה משניהם | |||||||||||||||||||||||||||||||||||||||||||||||||||||
| The arithmeticians carry out this division in two ways: | והנה החלוקה הזאת עושים אותה בעלי החשבון על שני דרכים | |||||||||||||||||||||||||||||||||||||||||||||||||||||
| The first way is as mentioned by the late R. Abraham Ibn Ezra the wise. | הדרך האחת כאשר הזכירה החכם ר' אברהם ן' עזרא ז"ל | |||||||||||||||||||||||||||||||||||||||||||||||||||||
| The second way is called by the Gentiles "galea" [galley] in their language and its meaning is a boat. | והדרך השנית היא אשר קורין אותה הלועזים גליאה בלשו' ופירושו דוגית | |||||||||||||||||||||||||||||||||||||||||||||||||||||
| I will mention both ways here. | ואני אזכיר הנה גם שתי הדרכים | |||||||||||||||||||||||||||||||||||||||||||||||||||||
| The first way is when you want to divide a number by a number, whether one by many, many by one, or many by many. | הדרך הראשון הוא כאשר תרצה לחלק מספר על מספר בין שיהיה אחד על רבים או רבים על אחד או רבים על רבים | |||||||||||||||||||||||||||||||||||||||||||||||||||||
| First, write the number that you want to divide. | תכתוב המספר אשר רצית לחלקו ראשונה | |||||||||||||||||||||||||||||||||||||||||||||||||||||
| In integers, the dividend must always be greater than the divisor. | וראוי שיהיה לעולם [המספר המתחלק][47] יותר מהמחולק עליו בשלמים | |||||||||||||||||||||||||||||||||||||||||||||||||||||
| Then, write the number, by which you want to divide it, in the bottom line, and leave a space between the upper line and the bottom line, in order to write between them the result of the division. | אחר תכתוב המספר אשר תרצה לחלק עליו בטור השפל [וריוח תשים בין העליון והשפל][48] כדי שתשים ביניהם העולה בחלוק | |||||||||||||||||||||||||||||||||||||||||||||||||||||
| Beware that you write the bottom number with its ranks corresponding to the ranks of the upper line, each facing its corresponding. | והשמר שתכתוב המספר השפל במדרגותיו כנגד מדרגות הטור העליון כל אחד כנגד הנכחי לו | |||||||||||||||||||||||||||||||||||||||||||||||||||||
| Start dividing from the highest rank. Consider it as units and divide it by the highest rank of the bottom line. | אחר תתחיל לחלק מהכלל היותר גדול ותחשבהו כאלו הם אחדים ותחלקהו על הכלל היותר גדול שבטור השפל | |||||||||||||||||||||||||||||||||||||||||||||||||||||
| If the highest rank of the upper line, when you consider it as units, is less than the highest rank of the bottom line, shift it back, so it will be greater than the highest rank of the bottom line and divide by it. | ואם הכלל היותר גדול שבטור העליון כשתחשבהו במספר האחדים יהיה יותר מעט מהכלל שבטור השפל החזירהו אחורנית ואז יהיה יותר גדול מהכלל שבטור השפל ותחלק עליו | |||||||||||||||||||||||||||||||||||||||||||||||||||||
| See, if the number by which you divide is in the first rank, write the result of division in the last rank; if it is in the second rank, write the result of division in the second rank backwards, i.e. the second from the last; and so on in that order. | וראה ואם המספר אשר תחלק עליו הוא מהמדרגה הראשונה כתוב העולה בחלוק במדרגה האחרונה ואם הוא במדרגת השנית כתוב העולה בחלוק במדרגה השנית אחורנית ר"ל השנית לאחרונה וכן על זה הסדר | |||||||||||||||||||||||||||||||||||||||||||||||||||||
| If any number remains in the ranks of the first line that cannot be divided in its rank, shift it back and consider it as ten, i.e. any of the remainders that cannot be divided, and add it to the closest lower rank, then divide it. | ואם ישאר שום מספר בכללי הטור הראשון אשר לא יכול להתחלק במדרגתו השיבהו אחורנית וחשבהו עשרה ר"ל כל אחד מהנשארים אשר לא יכול להתחלק וחברהו עם המדרגה הקרובה לו השפלה ממנו וחלקהו | |||||||||||||||||||||||||||||||||||||||||||||||||||||
| Do the same if there is a remainder from this rank as well, and so on until you reach the rank of the units. | וכן אם ישאר גם מזאת המדרגה ככה תעשה עד שתגיע למדרגת האחדים | |||||||||||||||||||||||||||||||||||||||||||||||||||||
| You should also know that if the digits by which you divide are numerous, and when you divide the rank of the first line by the rank of the second line nothing remains, you should not give it all the parts, but leave one part, in order to divide it by the rest of the digits in the bottom line and the same for every rank. | וכן ראוי שתדע שאם היו המספרים אשר תחלק עליהם רבים וכשחלקת הכלל שבטור הראשון על הכלל שבטור השני לא נשאר דבר אין ראוי שתתן לו [כל][49] החלקים אבל תשאיר חלק אחד כדי שתשיבהו אחורנית ותחלקהו גם על שאר המספר שבטור השפל וכן בכל מדרגה ומדרגה | |||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון רצינו לחלק ארבעת אלפים ומאתים [50]וחמשה עשר על ארבעה עשר | |||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כזאת הצורה | |||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקנו הד' שבטור העליון שהוא במדרגת האלפים על האחד שבטור השפל שהוא במדרגת העשרות ונתנו לו שלשה כדי שנוכל לחלק גם על הארבעה האחדים ואלה השלשה כתבנום במדרגה השנית אחורנית תחלתה מהכללים לפי שהאחד אשר חלקנו עליו היה במדרגה השנית מהאחדים | |||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד האחד הנשאר השיבונוהו אחורנית לעשרה וחברנוהו עם השני אשר במדרגת המאות ונהיו י"ב חסרנו מהם הד' אחדים שלשה פעמים ולא נשאר דבר להיותו מקושר וכן עדין לא יצא לחוץ כתבנו ספרא אחרי הג' במדרגת העשרות | |||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד שבנו לחלק ט"ו שבטור העליון על הי"ד שבטור השפל ונתננו לו אחד וכתבנוהו במדרגת האחדים במדרגה הראשונה מפני שגם המחולקי' הם במדרגה הרביעית ממדרגת האלפים ונשאר אחד בלתי מתחלק | |||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומזה ידענו שהחלק הוא שלש מאות ואחד ונשאר גם אחד אשר לא נחלק | |||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון אחר רצינו לחלק מאת אלף וארבעת אלפים וספרא ושלשים וארבעה על קי"ד | |||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כזה | |||||||||||||||||||||||||||||||||||||||||||||||||||||
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
השיבונוהו האחד שבטור העליון שהוא במדרגת העשר רבבות אל עשרה אחורנית במדרגת הרבבות ונתננו לחלק תשעה הוצאנו תשעה פעמים אחד ממנו נשאר אחד ואלה התשעה כתבנום במדרגה השלישית אחורנית מן הכלל המתחלק כי חלקנום על אחד שהוא במדרגה השלישית מן האחדים | |||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והאחד אשר נשאר במדרגת הרבבות השבנוהו אחורנית עשרה במדרגת האלפים וחברנוהו עם הארבעה אשר שם ונהיו י"ד גרענו עוד מהם תשעה פעמים אחד שהוא במדרגת העשרות ונשארו ה' במדרגת האלפים | |||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד גרענו מה0' ד' פעמים תשעה שהם האחדים שבטור השפל והם ל"ו ולא הספיקו השיבונו הד' אחורנית לעשרות שהם במדרגת המאות ונהיו מ' גרענו מהם הל"ו נשארו ד' במדרגת המאות וא' במדרגת האלפים | |||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד שבנו לחלק הא' שבמדרגת האלפים והד' שבמדרגת המאות על הקי"ד שבטור השפל ונתננו אחד לחלק וכתבנוהו במדרגה השנית אחרי הט' | |||||||||||||||||||||||||||||||||||||||||||||||||||||
|
גרענו א' מהא' אשר במדרגת האלפי' ולא נשאר דבר במדרגת האלפים | |||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד גרענו הא' אשר במדרגת העשרות מהארבעה אשר במדרגת המאות נשארו ג' במדרגת המאות | |||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד גרענו הד' האחדים שבטור השפל מהג' שבמדרגת המאות ולא הספיקו ולכן החזרנו הא' אחורנית לעשרה וחברנום עם הג' שבמדרגת העשרות ונהיו י"ג במדרגת המאות נשארו ב' גרענו הארבעה מהי"ג נשארו ט' במדרגת העשרו' | |||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד שבנו לחלק הב' אשר נשארו במדרגת המאות והט' אשר נשארו במדרגת העשרות על הקי"ד ונתננו ב' לחלק וכתבנוהו במעלת האחדים | |||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והוצאנו ב' פעמים א' מהב' ולא נשאר דבר | |||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד הוצאנו ב' פעמים א' [מהט'][51] ולא נשארו במדרגת העשרות רק ז' | |||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד הוצאנו ב' פעמים ד' מהד' שבטור העליון ולא הספיק ולכן החזרנו א' מהז' שהיו על מדרגת העשרו' אחורנית לעשר וחברנום עם הד' שבטור העליון ונהיו י"ד וגרענו מהם הח' ונשארו ו' במדרגת האחדים וו' במדרגת העשרות | |||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וידענו מזה שנחלק מספרנו לתשע מאות וי"ב חלקים [52]ונשארו גם ס"ו אשר לא נחלקו | |||||||||||||||||||||||||||||||||||||||||||||||||||||
| You should know that when you divide the numbers in the upper line by all the numbers in the bottom line, before [the procedure] is complete, if not enough remains there to be divided by the following [digits of the quotient], you should write a zero next to the [digits of the quotient] by which you have divided. Then, when you start dividing by the following [digit], write it next to the zero, as we did in the first example above. | וראוי לך לדעת שכאשר תחלק המספרים שבטור העליון על כל המספרים שבטור השפל וקודם שתצא לחוץ הותרו ולא היה לשם השארות שיקשרו עם הבאים אחריהם אז ראוי לך להשים בצד החלקים שחלקת סיפרא ואחרי כן כשתתחיל לחלק הבאים תכתבם בצד הסיפרא כאשר עשינו בדמיון הראשון אשר למעלה מזה | |||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון אחר רצינו לחלק חמשת אלפים ומאתים וארבעה עשר על מאה וסיפרא ושמנה | |||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כזה | |||||||||||||||||||||||||||||||||||||||||||||||||||||
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נתננו לחלק ד' כדי שיספיקו וכתבנוהו במדרגה השלישי' אחורנית כי האחד אשר חלקנום עליו היה במדרגה השלישית ממדרגת האחדים | |||||||||||||||||||||||||||||||||||||||||||||||||||||
|
גרענו ארבעה פעמים אחד ממנו ונשאר א' במדרגת האלפים | |||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד גרענו ד' פעמים שמנה שהם ל"ב מהא' ולא הספיק והשיבונוהו אחורנית לעשרות ונהיו י"ב כי חברנום עם הב' שבמדרגת המאות ועדין אינו מספיק להוציא מהם ל"ב השיבונו אחורנית הד' מהי"ב לעשרות וחברנום עם הא' אשר במדרגת העשרות ונהיו מ"א ונשארו במדרגת המאות ח' וגרענו הל"ב ממ"א ונשארו ט' במדרגת העשרות | |||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד שבנו לחלק השמנה על הא' ונתנו לו ח' וכתבנום במדרגה הראשונה מהאחדים | |||||||||||||||||||||||||||||||||||||||||||||||||||||
|
גרענו ח' מח' | |||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד גרענו ח' פעמים ח' שהם ס"ד מהט' שהם במדרגת העשרות ולא הספיקו ולכן השיבונו אחורנית הו' אל האחדים ונהיו ס' וחברנום עם הד' שבטור העליון ונהיו ס"ד וגרענו מהם הס"ד ונשארו ג' במדרגת העשרות | |||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וידענו מזה שהחלק הוא מ"ח ונשארו גם שלשים אשר לא נתחלקו | |||||||||||||||||||||||||||||||||||||||||||||||||||||
| This is one of the two ways of division. | זאת היא הדרך האחת מהשתי דרכים מדרכי החלוקה | |||||||||||||||||||||||||||||||||||||||||||||||||||||
| Galea | ||||||||||||||||||||||||||||||||||||||||||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| The second way, which is called in their language "galea" [galley], is the way most traders use. | הדרך השנית והיא אשר תקרא בלשונם גליאה היא דרך שרוב הסוחרים משתמשים בה | |||||||||||||||||||||||||||||||||||||||||||||||||||||
| It is that you write the number you wish to divide in the upper line and the number, by which you want to divide it, in the bottom line. | והוא שתכתוב המספרים אשר תרצה לחלק אותם בטור העליון והמספרים אשר תרצה לחלק עליהם בטור השפל | |||||||||||||||||||||||||||||||||||||||||||||||||||||
| Yet, you do not write its ranks corresponding to the upper [ranks], but you write its highest rank, whichever it may be, under the highest rank in the first line and the digit that follows corresponding to the digit that is next to the highest. | ולא תכתבם במדרגתם כנגד העליונים אבל איזו מדרגה שיהיו כתב הכולל שלהם תחת הכולל שבטור הראשון והמספר הבא אחריו כנגד המספר אשר בצד הכולל | |||||||||||||||||||||||||||||||||||||||||||||||||||||
| Then, you start dividing the highest rank in the upper line by the highest rank in the bottom line, but you do not give it all that you find: | אחר תתחיל לחלק הכולל שבטור העליון על הכולל שבטור השפל ולא תתן לו כל מה שתמצא | |||||||||||||||||||||||||||||||||||||||||||||||||||||
| If the digits next to it are not enough to be divide by the digits, by which you divide, leave one, or more, as much as you need and see how many times it is found in it, i.e. the highest rank in the other highest rank, after you leave [something] of it, if you leave; write the number of times it is found in it in a special place. | ואם אין המספרים אשר בצדו מספיקים לחלקם על המספרים אשר תחלק עליהם תשאיר אחד או יותר לפי מה שתצטרך ותעיין כמה פעמים הוא נכנס בו ר"ל הכולל בכולל אחר שהשארת ממנו אם השארת וכמספר הפעמים אשר יכנס בו כתוב במקום מיוחד המספר ההוא | |||||||||||||||||||||||||||||||||||||||||||||||||||||
| Multiply the highest rank of the number, by which you divide, by the number that is written in the special place and subtract [the product] from the highest rank of the dividend; write the remainder above it, if [something] remains and erase the former. | ותכה עם המספר הזה הכתוב במקום המיוחד את הכולל מן המספרים אשר תחלק עליהם ותגרעהו מכולל המספר הנחלק והנשאר אם ישאר כתוב אותו למעלה ממנו ותמחוק האחר | |||||||||||||||||||||||||||||||||||||||||||||||||||||
| Multiply also the digit that comes after the highest rank of the number, by which you divide, by the number that is written in the special place, subtract [the product] from the digit that comes after the highest rank of the dividend, and proceed according to the rule. | עוד תכה עם המספר הכתוב במקום המיוחד את המספר הבא אחר הכולל מהמספר אשר תחלק עליו ותגרעהו מהמספר הבא אחר הכולל מהמספרים הנחלקים ותעשה כמשפט | |||||||||||||||||||||||||||||||||||||||||||||||||||||
| Do the same until you divide by all the digits [of the number], by which you divide. | וכן תעשה עד שתחלק על כל המספרים [53]אשר תחלק עליהם | |||||||||||||||||||||||||||||||||||||||||||||||||||||
| If the divided digit is not enough to subtract from it, shift back the remaining one, or more, if there is any, as tens, and divide them by the number, by which you divide. Do this until you subtract all the digits, by which you divide. | ואם לא הספיק המספר הנחלק לגרוע ממנו תחזיר את האחד הנשאר או היותר אם היו אחורנית לעשרות ותחלקם על המספר אשר תחלק עליהם וכן תעשה עד שתוציא כל המספרים הנחלק עליהם בחלוקה | |||||||||||||||||||||||||||||||||||||||||||||||||||||
| Write again the digits, by which you divide: go down one rank, start from there and write [them] in order. | עוד תשוב לכתוב המספרים אשר תחלק עליהם ותרד מדרגה אחת ותתחיל משם ותכתוב כסדר | |||||||||||||||||||||||||||||||||||||||||||||||||||||
| See how many times the digit in highest rank by which you divide is found in the second digit that comes after the highest rank of the dividend, and write that number, i.e. the number of times, next to the number you wrote in the special place. Multiply by it all the digits, by which you divide, and proceed according to the rule. Subtract the product of the digits, by which you divide, from the first remaining digit of the dividend, erase the written digit and write what remains above it. | ותראה כמה פעמים הוא נכנס הכולל מהמספרים אשר תחלק עליהם במספר השני הבא אחר הכולל במספרים הנחלקים והמספר ההוא ר"ל כמות הפעמים כתבהו בצד המספר אשר כתבת במקום המיוחד והכה עמו כל המספרים אשר תחלק עליהם ועשה כמשפט ותוציא הנכפל מהמספרים אשר תחלק עליהם מהראשון שבמספרים המחולקים הנשארים ותמחוק המספר הכתוב ואשר ישאר כתבהו עליו | |||||||||||||||||||||||||||||||||||||||||||||||||||||
| Do this until everything is gone. | כן תעשה עד שתצא בחוץ | |||||||||||||||||||||||||||||||||||||||||||||||||||||
| If, before everything is gone, you have subtract all the digits by which you divide from those digits [of the dividend] and nothing remains, write a zero outside, where you write the quotient, next to the present [digit of] the quotient. Then, divide again the next digits, until everything is gone and write [the following digits of] the quotient next to the zero. | ואם בתוך המספר קודם שתצא בחוץ הוצאת כל המספרים אשר חלקת עליהם מהמספרים ההם ולא נשאר דבר כתוב סיפרא בחוץ לשם שאתה כותב החלקים בצד החלק אשר אתה בו עוד תשוב לחלק המספרים הבאים עד שתצא בחוץ והחלקים כתבם בצד הסיפרא | |||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון רצינו לחלק ד' אלפים ומאתים וט"ו על י"ד | |||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כזאת הצורה | |||||||||||||||||||||||||||||||||||||||||||||||||||||
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כתבנו אותם בטור העליון והי"ד בטור השפל | |||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והתחלנו מהמדרגה היותר כוללת ושמנו גם קו אחד יורד בצד המספרים וראינו כמה פעמים נכנס א' בד' ומצאנו שנכנס ד' פעמים ולא נתננו לו כל הד' אבל נתננו לו ג' כדי שיספיק לחלק גם על השאר והג' כתבנום בחוץ בצד הקו היורד | |||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וגרענו הא' ג' פעמים מהד' ונשאר א' ומחקנו הד' וכתבנו למעלה במדרגתו א' | |||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הוצאנו הד' ג' פעמים שהם י"ב מזה האחד הנותר אחר שהשיבונוהו אחורנית לעשרה וחברנוהו עם הב' ונהיו י"ב ולא נשאר דבר ומפני שלא נשאר דבר קודם שנצא בחוץ כתבנו בצד הג' סיפרא | |||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד שבנו לחלק הט"ו שלמעלה על הי"ד ומחקנו הי"ד ממקומם וכתבנום תחת [הט"ו][54] כי הותירו ואינם קשורים אחר שיצאו ממקומם | |||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונתננו חלק אחד לאחד וכתבנוהו בצד הסיפרא ונשאר חלק א' בלתי מתחלק כי הוצאנו האחד מן האחד ומחקנו את שתיהם והוצאנו הד' מן הה' ונשאר א' ומחקנו את שתיהן וכתבנו האחד למעלה | |||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וידענו מזה שנחלק לשלש מאות וסיפרא וחלק א' ונשאר גם א' בלתי מתחלק | |||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון אחר רצינו לחלק מאת אלף וסיפרא וארבעת אלפים וסיפרא ושלשים וארבעה על קי"ד | |||||||||||||||||||||||||||||||||||||||||||||||||||||
| כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
השיבונו האחד שבמספרי הטור העליון הכולל לעשרות וכתבנו אותו במדרגת הרבבות ולכן כתבנו הקי"ד ממנו כי הוא לא הספיק | |||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וראינו שהמספר אשר נחלק עליו נכנס [בו][55] תשעה פעמים וכתבנו התשעה בחוץ בקו היורד בחוץ | |||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפלנו האחד עם התשעה ונהיו ט' גרענו אותם מן העשרה שבמדרגת הרבבות נשאר א' במדרגת הרבבות ומחקנו [56]האחד שבטור השפל | |||||||||||||||||||||||||||||||||||||||||||||||||||||
|
[עו' כפלנו הט' עם הא' השני שבטור השפל][57] ונהיו ט' החזרנו אחורנית את האחד שבמדרגת הרבבות לעשרו' וחברנוהו עם הארבעה שיש שם במדרגת האלפים [ונהיו י"ד גרענו מהם הט' ונשארו ה'][58] ומחקנו הא' והד' וכתבנו על מדרגת האלפים ה' | |||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד כפלנו הד' שבטור השפל על הט' ונהיו ל"ו והחזרנו הד' מהה' שבמדרגת האלפי' לעשרות על מדרגת המאות ונשאר אחד במדרגת האלפים ומחקנו הה' וכתבנו במקומו א' והד' אשר החזרנו נהיו ארבעים וגרענו מהם הל"ו ונשארו ד' במדרגת המאות וכתבנום ומחקנו גם הד' בטור השפל | |||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומפני שהחשבון לא יצא בחוץ עדין שבנו עוד וכתבנו הקי"ד כל א' מהם קודם מדרגה אחת ממה שכתבנום בפעם הראשונה | |||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ורצינו לחלק הא' אשר בטור העליון שהוא במדרגת האלפים עם המספרים אשר בצדו עליהם וראינו שהם נכנסים בו פעם א' וכתבנו א' בחוץ בצד המספר אשר בצד הקו היורד בחוץ | |||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפלנוהו עם האחד שבטור השפל ונהיה א' גרענוהו מן האחד שבמדרגת האלפים ומחקנוהו וגם מחקנו גם זה האחד | |||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד כפלנו הא' שבחוץ עם הא' השני שבטור השפל וגרענוהו מהד' שבמדרגת המאות ונשארו שלשה ומחקנו הד' וכתבנו במקומם ג' וכן מחקנו גם הא' | |||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד כפלנו הא' שבחוץ עם הד' שבטור השפל ונהיו ארבעה רצינו לגרעם מן הג' הכתובים במדרגת העשרות ולא הספיקו ולכן השיבונו מן הג' שבמדרגת המאות אחד אחורנית לעשרות ומחקנום וכתבנו במקומם ב' והעשרות חברנום עם הג' שבמדרגת העשרות ונהיו י"ג גרענו מהם הד' נשארו ט' במדרגת העשרות ומחקנו הג' וכתבנו ט' במקומם וכן מחקנו גם הד' שבטור השפל | |||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד מפני שעדיין לא יצא החשבון בחוץ שבנו וכתבנו הקי"ד מדרגה אחת קודם המדרגה שכתבנום בפעם השנית | |||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וראינו כמה פעמי' הם נכנסים בחשבון הנשאר אשר לא נתחלק עדין ומצאנו שנכנסים ב' פעמים ולכן כתבנו בחוץ בצד הקו היורד מחוץ ב' | |||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפלנו אותם עם הא' שבטור השפל ונהיו ב' גרענום מהב' אשר במדרגת האלפים ולא נשאר דבר ומחקנו אותם וגם הא' שבטור השפל | |||||||||||||||||||||||||||||||||||||||||||||||||||||
|
[עוד כפלנו הב' עם הא' השני שבטור השפל][59] ונהיו ב' גרענום מהט' שבטור העליון הכתוב במדרגת העשרות ונשארו ז' מחקנו הט' וכתבנו ז' במקומם וכן מחקנו גם הא' השני שבטור השפל | |||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד כפלנו הב' עם הד' שבטור השפל ונהיו ח' ומפני כי לא הספיקו לגרעם מן הד' שבטור העליון במדרגת האחדים השיבונו מן הז' אחד אחורנית לי' ומחקנו הז' וכתבנו במקומם ו' והעשרה חברנום עם הד' ונהיו י"ד וגרענו מהם ח' ונשארו ו' ומחקנו את הד' וכתבנו במקומם ו' וכן מחקנו גם הד' שבטור השפל | |||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומזה ידענו שנחלקו לתשע מאות ושנים עשר חלק ונשארו גם ס"ו אשר לא נתחלקו | |||||||||||||||||||||||||||||||||||||||||||||||||||||
Check |
||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Scales by casting out nines [lit. by the Nine way]: | מאזנים על דרך התשעה | |||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תכפול מאזני המספר אשר [חלקת][60] עליו עם מאזני החלקים אשר כתבת אותם באמצע לפי הדרך הראשון ולפי הדרך השנית הם אשר כתבת אותם בצד הקו היורד בחוץ ואחר שתכפלם השליכם ט' ט' ואשר ישאר תשמור אותו | |||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה אם לא נשאר מהמספר אשר חלקת אותו שום דבר אשר לא נתחלק | |||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אבל אם נשאר קח גם המאזנים שלו וחבר אותם עם המספר [61]אשר שמרת ואם יעדיפו מהט' תשליך הט' והנשאר הוא השמור האמתי | |||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אחר תעיין גם אל מאזני המספרים שחלקת אותם ואם הם שוים עם השמור אפשר שחשבונך יהיה אמת ואם לאו דע בודאי שטעית | |||||||||||||||||||||||||||||||||||||||||||||||||||||
| Other scales by casting out sevens [lit. by the Seven way]: | מאזנים אחרים על דרך הז' | |||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תחשוב כללי המספר אשר חלקת עליהם אלו עם אשר בצדם כאלו הם עשרות ואחדים והשליכם ז' ז' ואשר נשאר תחשבהו עוד לעשרות והמספר אשר בצדו לאחדים והשליכם ז' ז' עד שתגיע למדרגת האחדים ואשר ישאר יקרא מאזני המספר אשר חלקת עליו | |||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד כן תעשה במאזני החלקים והם אשר כתבתם באמצע בדרך הראשונה ובחוץ בצד הקו היורד בדרך השנית והשליכם ז' ז' ואשר ישאר יקרא מאזני החלקים | |||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אחר תכפול מאזני המספר אשר חלקת עליו על מאזני החלקים ואשר יהיה השליכהו ז' ז' ואשר ישאר שמרהו ויקרא השמור | |||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה אם לא נשאר במספרים אשר חלקת אותם מספר אשר לא נתחלק | |||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אכן אם נשאר מספר אשר לא נתחלק קח מאזני המספר ההוא ג"כ בכזה הדרך והשליכהו ז' ז' והנשאר חברהו עם השמור ואז יקרא השמור באמת | |||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אחר תעיין אל מאזני המספרים אשר חלקת אותם וזה ג"כ כשתוציאם בזה הדרך והשליכם ז' ז' ואשר ישאר אם הוא שוה לשמור באמת אפשר שיהיה חשבונך אמת ואם לאו דע בודאי שטעית | |||||||||||||||||||||||||||||||||||||||||||||||||||||
| Other scales that are scales of truth: | מאזנים אחרים שהם מאזני צדק | |||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תכפול החלקים אשר באמצע בדרך הראשונה או בצד הקו היורד בחוץ בדרך השנית עם המספר אשר חלקת עליו ואשר יהיה אם הוא שוה עם המספרים אשר חלקת אותם אם נחלקו הכל או אם לא נחלקו הכל אם הוא שוה להם אחר אשר תגרע מהם המספרים אשר לא נחלקו אז תדע בודאי כי חשבונך אמת ואם אין דע בודאי שטעית |
Chapter Two: To Sum Up a Number with a Number Successively or by another Sequence [= Progression] |
הפרק השני להוסיף מספר על מספר כסדרו או על סדר אחר |
| Whoever wants to sum up a number with a number successively, multiplies it by its half plus one half and the result is the required.
|
הרוצה להוסיף מספר על מספר כסדרו יכפול אותו על חציו בתוספת חצי אחד והעולה הוא המבוקש |
|
דמיון רצינו להוסיף המספרים שהם מא' עד י' [זה על זה][62] |
|
כפלנו הי' בה' ונהיו נ' עוד הוספנו ה' שהוא חצי י' ונהיו נ"ה וזהו המבוקש |
|
דמיון אחר לנפרדים רצינו לדעת כמה תוספת המספרים זה על זה מא' עד ט' |
|
והנה חציו ד' וחצי הוספנו על זה עוד חצי אחר ונהיו ה' כפלנו את הט' בה' ונהיו מ"ה וזהו המבוקש |
| All numbers are according to these two examples. | ועל אלו שני הדמיונים כל המספרים |
| If you want, square the whole number, add to it its root, then take its half and this is the required.
|
ואם תרצה תרבע כל המספר ותוסיף עליו שרשו וקח מחציתו והוא המבוקש |
|
דמיון כפלנו הי' על עצמו ונהיו ק' הוספנו עליו שרשו שהוא י' ונהיו ק"י וחציים נ"ה וזהו המבוקש |
|
| |
| Said Mordecai: I have found another very suitable way, which is that you multiply the last required number by the number that follows it, and half the product is the required.
|
אמר מרדכי [מצאתי][63] דרך אחרת נכבדת מאד והוא שתכה תכלית המספר המבוקש עם המספר הבא אחריו וחצי ההוה הוא המבוקש |
|
דמיון רצינו לדעת כמה תוספת המספרים שהם מא' עד י' |
|
הכינו הי' עם הי"א שהוא המספר הבא אחריו ונהיו ק"י וחציו נ"ה והוא המבוקש |
|
[64]שאלה אם ישאלך אדם חברתי מספרים והיו נ"ה כמה יהיו המספרים |
|
תשובה כפול המספרים הנ"ה על ב' וקח שרש המרובע שעבר וראה ואם הוא שוה למספר הנשאר מהמרובע שעבר כמוהם היו מספרים |
|
המשל כפלנו הנ"ה והיו ק"י והמרובע [שעבר][65] ק' ושרשו י' והמספר הנשאר מהמרובע שעבר שהוא ק' עד כפל הנ"ה שהם ק"י הוא י' ואם כן המספרים שחברת והיו נ"ה הם י' |
|
שאלה חברתי מד' עד י' כמה יהיו |
|
תשובה תעשה כמשפט הראשון ותמצאם נ"ה אחר תחבר מא' עד ג' ותמצאם ו' גרעם מנ"ה ונשארו מ"ט וזהו המבוקש |
| If someone asks you how much are the squares of the numbers up to a certain number successively: | ואם ישאלך אדם כמה הם [מרובעי][66] המספרים עד מספר פלוני על הסדר |
| Answer that he should find the sum of [the numbers themselves], then multiply it by two-thirds of the last number plus one third and the result is the required.
|
תשיב שימצא חבורם בפשוטיהם ואחר יכה אותם בשני שלישי תכלית המספר המבוקש עם תוספת שליש אחד ואשר יהיה הוא המבוקש |
|
דמיון כמה הם המרובעים המחוברים מא' עד ד' |
|
כפלנו אותם בחציים עם תוספת חצי אחד ונהיו י' עוד לקחנו שני שליש ארבעה עם תוספת שליש אחר והם שלשה הכינו אותם עם העשרה ונהיו שלשים וזהו המבוקש |
|
| |
Part Four: To Relate a Number To a Number |
החלק הרביעי לערוך מספר על מספר | |||||||||||||||||||||||||||||||
| In this chapter we will talk about integers because fractions have another way, which we will explain in the next chapter. | זה השער נדבר בו על שלמים כי לנשברים יש דרך אחרת נבאר אותה בפרק הבא | |||||||||||||||||||||||||||||||
| We say that although [the types of] proportions are ten, the ancients chose only three kinds of proportions that are: arithmetic proportion, geometric proportion and harmonic proportion. We will mention here only the geometric proportion, because it is needed for most of the questions that are asked in arithmetic. | ונאמר שעם היות שהאמצעיים הם עשרה אבל הקדמונים לא בחרו רק שלשת מיני הערכים והם ערכי החשבון וערכי המדות וערכי הנגונים ואנחנו לא נזכיר הנה רק ערכי המדות כי הם צורך לרוב השאלות הנעשות בחשבון | |||||||||||||||||||||||||||||||
| It has already been clarified in geometry that proportion is not possible with less than three terms. Even if the mean term is represents two terms, a proportion occurs with four terms. | וכבר התבאר בחכמת השעור כי התיחסו' לא תתכן בפחות משלשה גבולים ואם הגבול האמצעי הוא בעד שני גבולים התיחסות תפול בד' גבולים | |||||||||||||||||||||||||||||||
| Therefore, for all the numbers, such that the ratio of the first to the second is as the ratio of the third to the fourth, if you sum the squares of the four of them, it is equal to [the sum of] the square of the first and the fourth summed together and the square of the difference between the second and the third. | מפני זה כל המספרים אשר יחס הראשון אל השני כיחס השלישי אל הרביעי אם תחבר מרובע ארבעתם יהיה שוה למרובע הראשון והרביעי מחוברים ומרובע ההפרש שיש בין השני והשלישי | |||||||||||||||||||||||||||||||
| Also, if you sum the square of the second and the third together with the square of the difference between the first and the fourth it is equal to this number. | וכן אם תחבר מרובע השני והשלישי יחד עם מרובע ההפרש שיש בין הראשון לרביעי יהיה שוה בחשבון הזה | |||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||
| Similarly, when the proportional terms are switched, because, the ratio of the first to the third is as the ratio of the second to the fourth.
|
וכן כאשר הומרו היו מתיחסים כי יחס הראשון אל השלישי יהיה כיחס השני אל הרביעי | |||||||||||||||||||||||||||||||
| Therefore, from the three knowns we know the fourth unknown. | ומפני זה מהשלשה הידועים נדע הרביעי המוסכל | |||||||||||||||||||||||||||||||
| The arithmeticians always tend to write the unknown at the last end, whether it is [one] of the means, or it is the first extreme, provided that one knows how to arrange the others. | וכבר נהגו בעלי החשבון לכתוב המוסכל בקצה האחרון לעולם בין שיהיה מהאמצעיים ובין שיהיה הקצה הראשון ובלבד שידע איך יסדר האחרים | |||||||||||||||||||||||||||||||
|
דמיון אם שאל שואל שאם השמנה ירויחו ד' הו' מה ירויחו | |||||||||||||||||||||||||||||||
|
הנה נקראו האמצעיים הד' והו' ושתי הקצוות השמנה והגלגל כזה | |||||||||||||||||||||||||||||||
| ||||||||||||||||||||||||||||||||
|
כפלנו שני האמצעיים ונהיו כ"ד חלקנום על הקצה הידוע שהם הח' ונהיה החלק ג' ומזה ידענו שבמקום הגלגל צריך ג' והוא המבוקש | |||||||||||||||||||||||||||||||
|
וכן אם הפך הסדר ושאל אם [67]הד' הרויחו אותם הח' מהו אשר ירויחו הו' | |||||||||||||||||||||||||||||||
|
ועתה נהיו שני האמצעיים השמנה והגלגל כזה | |||||||||||||||||||||||||||||||
| ||||||||||||||||||||||||||||||||
| When we multiply the two extremes and divide by the known mean, we find the unknown mean. | והנה כשנכפול שתי הקצוות ונחלקהו על האמצעי הידוע נמצא האמצעי המוסכל | |||||||||||||||||||||||||||||||
|
כפלנו ד' על ו' ונהיו כ"ד חלקנום על ח' ונהיה החלק ג' וזהו המבוקש | |||||||||||||||||||||||||||||||
|
וכן אם הפך השאלה ושם הגלגל ראשונה ואמר מה ירויחו הו' אם הח' ירויחו ארבעה | |||||||||||||||||||||||||||||||
|
כזה | |||||||||||||||||||||||||||||||
| ||||||||||||||||||||||||||||||||
| We multiply the known extreme by the mean that is next to the unknown, then divide by the mean that is next to the known extreme and we find the unknown. | נכפל הקצה הידוע עם האמצעי אשר בצד המוסכל ונחלקהו על האמצעי אשר בצד הקצה הידוע ונמצא המוסכל | |||||||||||||||||||||||||||||||
| Even though all of these are the right way, the arithmeticians always make the zero the last extreme, provided that they keep order, so that those who yield the profit are in their place and the profit is in its place. | ואע"פ שכל אלה הם דרך נכונה אבל לעולם בעלי החשבון יהפכו הגלגל אל הקצה האחרון בלבד שישמרו הסדר עד שיהיו המרויחים במקומם והריוח במקומו | |||||||||||||||||||||||||||||||
| Also, if there were measures and dinars, the dinars are in their place and the measures in their place - one should be careful not to replace them, because he would be wrong. | וכן אם היו מדות ודינרים שיהיו הדינרים במקומם והמדות במקומם ויזהר שלא יחליף כי יטעה | |||||||||||||||||||||||||||||||
|
דמיון אם שאל שואל שאדם אחד מוכר חטה ז' מדות בי"ב פשוטים כמה מדות יתן בי"ד פשוטים | |||||||||||||||||||||||||||||||
|
ראוי להפך השטה לבד שישמור הסדר ושיכתוב כך אם י"ב פשוטים נתנו ז' מדות הי"ד פשוטים מה יתנו | |||||||||||||||||||||||||||||||
|
ואז נופל הגלגל בתכלית האחד ולעולם בזה הסדר | |||||||||||||||||||||||||||||||
| ||||||||||||||||||||||||||||||||
| One multiplies the means and divide [the product] by the first extreme, then he deduces the last extreme. | הוא כופל האמצעיים ומחלק אותם על הקצה הראשון ולומד על הקצה האחרון | |||||||||||||||||||||||||||||||
| When you divide the product by the divisor and it is not reduced, but a number remains undivided, see, if this undivided number counts the number by which it is divided, know that it is its part as many times as it counts it: if it counts it twice, it is its half; if thrice, it is its third; and so on. | וכאשר תחלק הכפול על הנחלק עליו ולא יצא בצמצום אבל נשאר חשבון אשר לא נתחלק [תעיין ואם זה החשבון אשר לא נתחלק][68] הוא תחת כפלי החשבון אשר נתחלק עליו דע כי הוא חלק ממנו לפי הפעמים אשר ישערהו כי אם ישערוהו ב' פעמים הוא מחציתו ואם בג' פעמים הוא שלישיתו וכיוצא בם | |||||||||||||||||||||||||||||||
| When it does not count it, if you want, extract the part that counts it, then decompose the remainder into fractions, or, if you want, decompose all into fractions, then divide. If there is still a remainder, decompose it into fractions of fractions, until it is reduced, or until something remains that is unperceivable. | ואם לא יהיה תחת כפליו אם תרצה תוציא ממנו חלק אשר יהיה תחת כפליו והשאר השיבהו אל פרטים או אם תרצה השב הכל אל הפרטים וחלקהו ואם ישאר עוד השיבהו אל פרטי פרטים עד שיצא בצמצום או עד שישאר דבר שאין חששא בו | |||||||||||||||||||||||||||||||
|
דמיון שאלנו אם הי"ב דינרים נתנו שבעה דינרים ריוח הי"ד [דינ'][69] כמה יתנו | |||||||||||||||||||||||||||||||
|
וכתבנו אותם כך | |||||||||||||||||||||||||||||||
| ||||||||||||||||||||||||||||||||
|
כפלנו האמצעיים ונהיו צ"ח חלקנום על הקצה האחד שהם י"ב ונהיה החלק הא' ח' ונשארו גם ב' אשר לא נתחלקו ומפני שאלה הב' הם תחת כפלי הי"ב וישערום בששה פעמים ידענו מזה שהמבוקש הוא ח' וששית | |||||||||||||||||||||||||||||||
|
אבל אם שאל אם הי"א דינרים נתנו [ז'][70] דינרים ריוח הי"ד מה יתנו | |||||||||||||||||||||||||||||||
|
כתבנום כך | |||||||||||||||||||||||||||||||
| ||||||||||||||||||||||||||||||||
|
כפלנו האמצעיים ונהיו צ"ח חלקנום על הי"א ונהיה החלק ח' ונשארו י' אשר לא נתחלקו השיבונו אותם אל פרטים שהם י"ב בדינר ונהיו ק"כ חלקנום אל י"א ונהיה [החלק הא' י' פרטים ונשארו עוד י' בלתי מתחלקים השיבונום אל פרטי פרטים שהם גם הם י"ב בפרוטה ונהיו ק"כ חלקנום על י"א ונהיה][71] החלק האחד י' ונשארו י' בלתי מתחלקים ומפני שאין בם חששא הנחנום וידענו מזה שהחלק הא' הוא ח' דינרים וי' פרוטות מי"ב בדינר וי' פרטי פרוטות מי"ב פרוטות ודבר שאין בו חששא וזהו הריוח אשר יתנו הי"ד דינרים | |||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||
| These are called "proportional triad" [lit. having three figures = rule of three]. | ואלה הם אשר יקראו אותם בעלי שלשת הצורות | |||||||||||||||||||||||||||||||
| There are, however, other problems, which are called "proportional pentad" [lit. having five figures], in which the one who asks adds one matter to each of the ratios, such as time etc. We write them as follows: | אולם יש שאלות אחרות אשר יקראו אותם בעלי חמש צורות והם אשר ישתף השואל דבר אחד עם כל אחד מהיחסים כגון זמן וכיוצא בו ואז נכתבם ככה | |||||||||||||||||||||||||||||||
|
דמיון אם אדם שכר ששה אנשים לעבוד עמו שלשה ימים בח' דינרים ועבדו עמו הארבעה בשני ימים כמה יקחו | |||||||||||||||||||||||||||||||
| Although there is no doubt that it consists of two proportions of proportional triad, the arithmeticians present a way to find all in one answer, in order to ease the one who calculates, as if it is one proportion, and they call it "proportional pentad" [lit. having five figures]. | אע"פ שאין ספק שזה מחובר משני ערכים אל שלש צורות אבל בעלי החשבון נתנו דרך למצא הכל בתשובה אחת כדי להקל על המחשב כאלו הוא ערך אחד וקראו אותו בעל חמש צורות | |||||||||||||||||||||||||||||||
|
ונעשה ככה | |||||||||||||||||||||||||||||||
| ||||||||||||||||||||||||||||||||
|
[72]אנו כופלים את הקצה הראשון עם הימים אשר בצדו ומה שיהיה הוא היסוד אשר אנו חולקים עליו את השאר כאשר נראה | |||||||||||||||||||||||||||||||
|
כפלנו הששה בשלשה ונהיו י"ח והוא היסוד אחר כפלנו הח' בד' ונהיו ל"ב עוד הל"ב בב' ונהיו ס"ד חלקנו [הס"ד][73] על הי"ח ונהיה החלק הא' ג' ונשארו גם י' בלתי מתחלקים השיבונו הי' אל הפרוטות אשר הם י"ב באחד ונהיו ק"כ חלקנום על הי"ח ונהיה החלק הא' ו' פרוטות ונשארו גם י"ב בלתי מתחלקים השיבונו הי"ב אל פרוטי פרוטות אשר הם י"ב באחד ונהיו קמ"ד חלקנום אל הי"ח ונהיה החלק הא' ח' וידענו מזה שהד' אנשים בשני ימים אשר עבדו יקחו ג' דינרים וו' פרוטות [וח' פרוטי פרוטות][74] לפי חשבון התנאי אשר התנה לעבוד עמו הו' אנשי' בשלשה ימים ולקחת ח' דינרים | |||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||
The Separate Part: Discusses Fractions |
החלק הנפרד המדבר על הנשברים | |||||||||||||||||||||||||||||||
| Said Mordecai: since the number is a discontinuous quantity and the fractions are continuous quantity, and the discontinuous and the continuous are two opposites, for one is endlessly subject to addition but not endlessly subject to division, because it stops [when reaching] one, while the other is endlessly subject to division, because the magnitude cannot be composed of parts that are indivisible. | אמר מרדכי להיות שהחשבון הוא מהכמה המתחלק והשברים הם שבר הכמה המתדבק והמתחלק עם המתדבק הם שני הפכים כי זה יקבל התוספת אל בלתי תכלית ולא יקבל החלוקה אל בלתי תכלית כי יעמוד באחד וזה יקבל החלוקה אל בלתי תכלית כי אי אפשר להיות הגודל מחובר מחלקים אשר לא יתחלקו | |||||||||||||||||||||||||||||||
| Therefore, the rules of fractions are the opposite of the rules of integers. | לכן היה משפטי השברים הפך משפטי השלמים | |||||||||||||||||||||||||||||||
| For, the product of integers is greater than the multiplicands, while the product of fractions is smaller than the multiplicands. | כי כפל השלמים הוא יותר מהמספרים אשר יכפלו [וכפל השברי' הוא פחות מהממספרי' אשר יכפלו][75] | |||||||||||||||||||||||||||||||
| Since one is mean between the integers and the fractions, its product is equal to itself, because it is drawn to both sides equally. | ומפני שהאחד הוא אמצעי בין השלמים ובין השברים לכן היה כפלו כמוהו כי ימשך אל שתי צדדיו בשווי | |||||||||||||||||||||||||||||||
| Since the magnitude of the fractions agrees with the parts that are summed in a whole unit, they borrowed their name, for the half is derived from two, the third from 3, the quarter from 4, so the denominator is derived from them, as we will explain. | ומפני שהשברים בגודל יסכימו עם החלקים שהם בכלל אחד מקובץ לקחו השם מהם כי החצי נגזר משנים והשלישית מג' והרביעית מד' ולכן יהיה המורה מהם כאשר נבאר | |||||||||||||||||||||||||||||||
| They are divided by the square of the denominator and the required is found. | אחר יחלקו על מרובע [המורה] [76] וימצא המבוקש | |||||||||||||||||||||||||||||||
| Multiplication of Fractions | ||||||||||||||||||||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
|
דמיון שאל השואל כפל שלישית על שלישית כמה יהיה | |||||||||||||||||||||||||||||||
|
והנה המורה ט' כי כפלנו ג' על ג' | |||||||||||||||||||||||||||||||
|
ומרבעו פ"א | |||||||||||||||||||||||||||||||
|
חלקנו המורה על מרובעו ועלה תשיעי' אחת ומזה ידענו שכפל שלישית על שלישית יהיה תשיעית | |||||||||||||||||||||||||||||||
|
וכן אם היו ב' שלישיות על ב' שלישיות | |||||||||||||||||||||||||||||||
|
המורה ט' כי כפלנו שלש על שלש הם ט' | |||||||||||||||||||||||||||||||
|
ומרובעו פ"א | |||||||||||||||||||||||||||||||
|
ומפני שהשלישיות היו ב' על ב' לקחנו בעד הב' שלישיות [ו' וכן ו' בעד הב' שלישיות][77] האחרים כפלנו ו' על ו' ונהיו ל"ו | |||||||||||||||||||||||||||||||
|
חלקנום על פ"א ונהיה החלק האחד שלישית ותשיעית וזהו המבוקש | |||||||||||||||||||||||||||||||
|
דמיון אחר השואל כמה ג' רביעיות הנכפלות על ארבע תשיעיות | |||||||||||||||||||||||||||||||
|
הנה המורה ל"ו כי כפל הארבעה על הט' הם ל"ו | |||||||||||||||||||||||||||||||
|
ומרבעו אלף רצ"ו | |||||||||||||||||||||||||||||||
|
ומפני כי הרביעיות היו ג' לקחנו י"ב בעדם כי ג' פעמים ד' הם י"ב ומפני שהתשיעיות הם ד' לקחנו בעדם ל"ו הכינו הי"ב עם הל"ו ונהיו תל"ב | |||||||||||||||||||||||||||||||
|
חלקנום על מרובע המורה שהם אלף רצ"ו ונהיה החלק האחד שלישית אחד ומזה ידענו שהמבוקש הוא שלישית אחד | |||||||||||||||||||||||||||||||
| However, the arithmeticians extract the denominator in an easier way, because this denominator is a large number, so they do like that: | אבל בעלי החשבון לוקחים המורה באופן יותר נקל כי זה המורה הוא רב החשבון ועושים ככה | |||||||||||||||||||||||||||||||
|
כשישאלו על כפל שלישית אחד על שלישית אחד כותבים האחד למעלה ואחר הם מתווכים עם קו וכותבים השלישית למטה וככה יעשו בשלישית האחרת כזאת הצורה | |||||||||||||||||||||||||||||||
| ||||||||||||||||||||||||||||||||
|
וכופלים האחד על האחד ונהיה אחד אחר כן יכפלו הג' על הג' ונהיו ט' אחר רואים מה ערך הא' אל הט' והוא המבוקש | |||||||||||||||||||||||||||||||
|
דמיון אחר כמה ב' שלישיות הכפולות על ד' רביעיות | |||||||||||||||||||||||||||||||
|
כתבנו הב' למעלה [78]והשלישיות למטה והתווכנו עם קו אחד וכן עשינו בד' הרביעיות כזה | |||||||||||||||||||||||||||||||
| ||||||||||||||||||||||||||||||||
|
כפלנו [הב' עם הד' ונהיו ח' עוד כפלנו][79] הג' שלמטה עם הד' שלמטה ונהיו י"ב אחר עייננו מה ערך הח' אל הי"ב והם שני שלישים וידענו מזה שהמבוקש הם שני שלישיות אחד | |||||||||||||||||||||||||||||||
| If we want to multiply integers and fractions by integers and fractions: | ואם רצינו לכפול שלמים ונשברים עם שלמים ונשברים | |||||||||||||||||||||||||||||||
| We write them like this: the integers and next to the them fractions; the same for those by which we want to multiply them. | אנו כותבים אותם ככה השלמים ובצדם הנשברים וכן אשר נרצה לכפול עליהם | |||||||||||||||||||||||||||||||
| Then, we decompose the integers to fractions as follows: we multiply the parts of the continuous by the integers as if they are also integers and add the number of the parts to them also, so they are all become fractions, like this: | אחר אנו משברים השלמים בכזה האופן אנו מכים חלקי המתדבק עם השלמים כמו שאם היו גם הם שלמים ואנו מחברים גם מספר החלקים עמהם ואז שבו כלם נשברים כזה | |||||||||||||||||||||||||||||||
|
כמה הם חמשה שלמים ושלשת רביעיות הכפולים על ד' שלמים וב' שלישיות | |||||||||||||||||||||||||||||||
|
והנה הצורה | |||||||||||||||||||||||||||||||
| ||||||||||||||||||||||||||||||||
|
הכינו הארבעה שהם מורה החלק המתדבק עם החמשה שלמים ושבו כ' חברנו גם הג' שהם מספר הרביעיות ונהיו כ' וג' רביעיות | |||||||||||||||||||||||||||||||
|
עוד הכינו הג' שהם מורה חלקי המתדבק עם הד' השלמי' ונהיו י"ב חברנו גם השנים עמהם ונהיו י"ד שלישיות | |||||||||||||||||||||||||||||||
|
והנה הראשונים כ"ג רביעיות ואלה י"ד שלישיות הכינו אלה עם אלה והיו שכ"ב | |||||||||||||||||||||||||||||||
|
עוד הכינו הד' עם הג' שהם מורי השלישיות והרביעיות ונהיו י"ב | |||||||||||||||||||||||||||||||
|
ראינו מה ערך השכ"ב אל הי"ב והם כ"ז פחות ששית וידענו מזה כי כפל ה' שלמים וג' רביעיות על ד' שלמים וב' שלישיות הם כ"ז פחות ששית | |||||||||||||||||||||||||||||||
| This is the method the arithmeticians use when there are fractions with integers, whether they are integers alone by integers and fractions, or integers and fractions by integers and fractions, or integers alone by fractions alone. | זאת היא הדרך אשר נהגו בעלי החשבון להתנהג בהיות שם שברים עם שלמים בין שהיו שלמים לבד עם שלמים ושברים ובין שהיו שלמים ושברים [עם שלימי' ושברי'][80] ובין שהיו שלמים לבד עם שברים לבד | |||||||||||||||||||||||||||||||
| When there are integers alone by fractions alone, there is also another way: that we take for the integers a denominator as their number, we multiply it by the numerator of the fraction, then divide it by the denominator of the fraction; the result is the required. | אמנם בהיות שלמים לבד עם שברים לבד יש גם כן דרך אחרת והיא שנקח בעבור השלמי' מורה כמספרם ונכה אותו עם מספר חלקי השברים ונחלקהו על המורה שנקח מהשברי' והעולה הוא המבוקש | |||||||||||||||||||||||||||||||
|
דמיון כמה הם ב' שלמים הכפולים על ג' רביעיות | |||||||||||||||||||||||||||||||
|
לקחנו בעד הב' שלמים ב' והוא המורה שלהם כפלנום עם ג' שהוא מספר חלקי השברים נהיו ו' חלקנום על ארבעה שהוא המורה שלקחנו מהשברים ונהיו אחד וחצי וזהו המבוקש | |||||||||||||||||||||||||||||||
| Proportions of Fractions | ||||||||||||||||||||||||||||||||
| If you want to relate fractions to fractions, whether there are integers with each of them, or with one of them, you should decompose the integers to fractions first and convert them to fractions according to the type of the fractions that are summed with them. | ואם רצית לערוך שברים על שברים בין שיהיו שלמים עם כל אחד מהם [או עם האחד][81] לבד ראוי בתחלה לשבור השלמים ולעשותם שברים כפי המין אשר היו השברים הדבקים עמהם | |||||||||||||||||||||||||||||||
|
וככה תכתבם | |||||||||||||||||||||||||||||||
| ||||||||||||||||||||||||||||||||
|
תכה בתחלה השני שלמים עם השלש שהוא מורה השלישיות ונהיו ו' אחר תחבר גם הב' עמהם שהוא מספר השלישיות ונהיו ח' שלישיות | |||||||||||||||||||||||||||||||
|
עוד הכינו הג' שלמים עם הה' שהוא מורה החמישיות ונהיו ט"ו חברנו גם הד' עמהם שהם מספר החמישיות ונהיו תשע עשרה חמישיות | |||||||||||||||||||||||||||||||
|
עוד הכינו הד' שלמים עם הו' שהם מורה הששיות ונהיו כ"ד חברנו גם הג' שהם מספר הששיות עמהם ונהיו כ"ז ששיות | |||||||||||||||||||||||||||||||
|
כי השאלה היתה אם הב' מדות וב' שלישיות יתנו לנו ג' דינרים וד' חמישיות הד' מדות ושלש ששיות כמה יתנו | |||||||||||||||||||||||||||||||
|
ושבה השאלה עתה אם הח' שלישיות יתנו לנו י"ט חמשיות הכ"ז ששיות כמה יתנו | |||||||||||||||||||||||||||||||
|
ואחר שהשיבונו השאלה אל השברים אנו כותבים אותם כך | |||||||||||||||||||||||||||||||
| ||||||||||||||||||||||||||||||||
|
אחר כן אנו מכים אותם כדמות מספריים | |||||||||||||||||||||||||||||||
|
ואנו מכים הח' עם הה' ונהיו מ' ואנו כותבים אותם [82]תחת הה' | |||||||||||||||||||||||||||||||
|
עוד אנו מכים המ' עם הו' ונהיו ר"מ ואנו כותבים תחת הו' וזהו המספר אשר נחלוק עליו | |||||||||||||||||||||||||||||||
|
עוד אנו מתחילים להכות הג' עם הי"ט ונהיו נ"ז ואנו כותבים אותם על הי"ט | |||||||||||||||||||||||||||||||
|
עוד אנו מכים הנ"ז עם הכ"ז ונהיו אלף תקל"ט | |||||||||||||||||||||||||||||||
|
אחר כן אנו מחלקים אותם על הר"מ ונהיו ו' שלמי' וב' חמישיות וחלק אחד משמנים וזהו המבוקש אשר יתן לנו החשבון הדרוש | |||||||||||||||||||||||||||||||
| Yet, if the problem involves proportional pentad, for instance, when the one who asks adds another thing to his question, like time etc., as we have explained for integers, then we write as follows: | אבל אם החשבון יהיה בעל חמש צורות כגון שישתף השואל דבר אחר עם שאלתו כגון זמן וכדומה לו כאשר בארנו בשלמים אז נכתוב ככה | |||||||||||||||||||||||||||||||
|
דמיון שאל השואל אם ב' רביעיות דינר בד' שמיניות היום שהם י"ב שעות כי שמינית היום ג' שעות הרויחו ג' רביעיות דינר הד' רביעיות בד' שמיניות היום כמה ירויחו | |||||||||||||||||||||||||||||||
|
וזה צורתו | |||||||||||||||||||||||||||||||
| ||||||||||||||||||||||||||||||||
|
אז נכפול הב' עם הד' שבטור העליון והם מספר הרביעיות והשמיניות ונהיו ח' | |||||||||||||||||||||||||||||||
|
אחר הכינו הח' עם הד' שבטור השפל ונהיו ל"ב וכתבנום תחתיהם | |||||||||||||||||||||||||||||||
|
עוד הכינו הל"ב עם הד' אשר בצדם שהם תחת הד' שבטור העליון ונהיו קכ"ח וכתבנום תחתיהם | |||||||||||||||||||||||||||||||
|
עוד הכינו הקכ"ח עם השמנה אשר בצדם ונהיו אלף כ"ד וכתבנום תחתיהם ואלה הם היסוד אשר נחלוק עליהם | |||||||||||||||||||||||||||||||
|
עוד אנו מכים הד' עם הח' שבטור השפל ונהיו ל"ב ואנו כותבי' אותם תחתיהם | |||||||||||||||||||||||||||||||
|
עוד אנו מכים הל"ב עם הג' שבטור העליון ונהיו צ"ו ואנו כותבים אותם עליהם | |||||||||||||||||||||||||||||||
|
עוד אנו מכים הצ"ו עם הד' אשר בצדם ונהיו שפ"ד ואנו כותבים אותם עליהם | |||||||||||||||||||||||||||||||
|
עוד אנו מכים השפ"ד עם הד' אשר בצדם ונהיו אלף תקל"ו ואנו כותבים אותם עליהם | |||||||||||||||||||||||||||||||
|
אחר כן אנו מחלקים אותם על האלף וכ"ד ויצא החלק אחד וחצי וידענו מזה שזהו החשבון המבוקש אשר ירויחו הד' רביעיות בד' שמיניות היום כי ירויחו א' וחצי דינר | |||||||||||||||||||||||||||||||
|
דמיון אחר לזה רצינו לדעת אם הב' שלישיות דינר בד' חמישיות היום ירויחו ו' שביעיות הח' תשיעיות בי' עשיריות כמה יתנו | |||||||||||||||||||||||||||||||
|
וכתבנום ככה | |||||||||||||||||||||||||||||||
| ||||||||||||||||||||||||||||||||
|
כפלנו הב' עם הד' שבטור העליון ונהיו ח' וכתבנום עליהם | |||||||||||||||||||||||||||||||
|
עוד הכינו הח' עם הז' שהוא שלישי לטור השפל ונהיו נ"ו וכתבנום תחת הז' שבטור השפל | |||||||||||||||||||||||||||||||
|
עוד הכינו הנ"ו עם הט' ונהיו תק"ד וכתבנום תחת הט' | |||||||||||||||||||||||||||||||
|
עוד הכינו התק"ד עם הי' ונהיו ה' אלפים ומ' וכתבנום תחת העשרה ואלה הם היסוד אשר נחלק עליהם | |||||||||||||||||||||||||||||||
|
עוד חזרנו והכינו את הג' עם הה' שבטור השפל ונהיו ט"ו וכתבנום למטה תחת הה' | |||||||||||||||||||||||||||||||
|
אחר הכינו את הט"ו עם הוו' שבטור העליון שהוא השלישי והיו צ' וכתבנום על הו' | |||||||||||||||||||||||||||||||
|
עוד הכינו הצ' עם הח' ונהיו שבע מאות וכ' וכתבנום על הח' | |||||||||||||||||||||||||||||||
|
עוד הכינו את השבע מאות וכ' עם הי' ונהיו ז' אלפים ומאתים | |||||||||||||||||||||||||||||||
|
חלקנו אותם על היסוד שהם ה' אלפי' מ' ונהיה החלק אחד ושליש וחלק א' מי' וידענו מזה שזהו מה שירויחו הח' תשיעיות דינר בי' עשיריות היום אם הב' שלישיות דינר הרויחו ו' שביעיות בד' חמישיות היום | |||||||||||||||||||||||||||||||
| This is the way all traders and arithmeticians use for these two types of fractions, i.e. the proportional triad and the proportional pentad. | זהו הדרך אשר ישתמשו בה כל הסוחרים ובעלי החשבון באלה שתי מיני הצורות לשברים ר"ל בבעלי השלש צורות ובעלי החמש צורות | |||||||||||||||||||||||||||||||
| However there is another way, but it requires dividing three times, and it is as follows: | אולם יש דרך אחרת גם היא נכונה אבל יצטרך לחלק שלש פעמים והיא זאת | |||||||||||||||||||||||||||||||
|
דמיון בבעלת [83]השלש צורות הראשונה שהשאלה היא אם הח' שלישיות יתנו לנו י"ט חמישיות הכ"ז ששיות כמה יתנו | |||||||||||||||||||||||||||||||
| We write them according to the first rule with a separating line. We multiply the second numerator in the upper line by the third and we divide [the product] by the first. We do the same with the bottom line. Then we divide the quotient of the upper line by the quotient of the bottom line; the result is the required. | אנו כותבים אותם כמשפט הראשון עם הקו המתויך כך אחר אנו מכים מספר החלקים שבטור העליון השני עם השלישי ואנו מחלקי' אותם על הראשון וכן נעשה גם בטור השפל אחר כן אנו מחלקים מה שיצא מן החלק בטור העליון על מה שיצא מן החלק בטור השפל והיוצא הוא המבוקש | |||||||||||||||||||||||||||||||
|
דמיון הכינו הי"ט עם הכ"ז ונהיו תקי"ג חלקנו אותם על הח' ונהיה החלק הא' ס"ד ושמינית ושמרנום | |||||||||||||||||||||||||||||||
|
עוד הכינו הה' עם הו' שבטור השפל ונהיו ל' חלקנו אותם על הג' ונהיה החלק האחד י' | |||||||||||||||||||||||||||||||
|
אחר כן חלקנו הס"ד ושמינית השמורים על הי' ונהיה החלק הא' ו' שלמים וב' חמישיות וחלק א' משמנים | |||||||||||||||||||||||||||||||
|
דמיון אחר על בעלת חמש צורות הראשונות אשר השאלה היא אם ב' רביעיות דינר בד' שמיניות היום הרויחו ג' רביעיות דינר בד' שמיניות היום כמה ירויחו ד' רביעיות | |||||||||||||||||||||||||||||||
|
וכתבנום כמשפט הראשון ככה | |||||||||||||||||||||||||||||||
|
כפלנו הב' על הד' שבטור העליון ונהיו ח' ושמרנום כי על אלה השמנה נחלק כפל המספרים הנשארים אשר בטור העליון | |||||||||||||||||||||||||||||||
|
אחר כן כפלנו הג' שבטור העליון על הד' שבטור השפל אשר תחתיו ונהיה י"ב עוד הכינו הי"ב עם הד' שבטור העליון אשר בצדם ונהיה מ"ח | |||||||||||||||||||||||||||||||
|
חלקנו אותם על הח' השמורים ונהיה החלק הא' ששה ושמרנום | |||||||||||||||||||||||||||||||
|
עוד כפלנו הד' על הח' שבטור השפל ונהיו שלשים ושנים ושמרנום כי על אלה הל"ב נחלק כפל המספרים הנשארים אשר בטור השפל | |||||||||||||||||||||||||||||||
|
[אח"כ כפלנו הד' על הד' אשר בטור השפל][84] בצדם ונהיו י"ו עוד כפלנו הי"ו עם [הח'][85] אשר בצדם ונהיו קכ"ח | |||||||||||||||||||||||||||||||
|
חלקנו אותם על הל"ב השמורים ונהיה החלק האחד ד' | |||||||||||||||||||||||||||||||
|
אחר כן חלקנו הששה אשר שמרנום על אלה הד' ונהיה החלק א' וחצי ומזה ידענו שהריוח אשר ירויחו הד' רביעיות דינר בד' שמיניות היום הוא אחד וחצי דינר אם הב' רביעיות בד' שמיניות הרויחו ג' רביעיות דינר | |||||||||||||||||||||||||||||||
| Addition of Fractions | ||||||||||||||||||||||||||||||||
| If you wish to sum up fractions with fractions: | ואם רצית לקבץ שברים עם שברים | |||||||||||||||||||||||||||||||
| You should extract their [common] denominator and see these fractions of the denominator, then relate them to the denominator and you will know how much is the sum. | אתה צריך לעשות מורה מהם ולהביט השברים ההם על אותו המורה ולערוך אותם על המורה ואז תדע כמה יהיה הסך | |||||||||||||||||||||||||||||||
|
דמיון שאל שואל קבצנו ב' שלישיות עם ג' רביעיות כמה יהיו | |||||||||||||||||||||||||||||||
|
הכינו הג' עם הד' ונהיו י"ב והוא המורה | |||||||||||||||||||||||||||||||
|
וב' שלישיותיו ח' | |||||||||||||||||||||||||||||||
|
וג' רביעיותיו ט' | |||||||||||||||||||||||||||||||
|
ושניהם מקובצים י"ז תערכם אל הי"ב שהוא האחד השלם יהיו א' שלם ושליש וחלק א' מי"ב | |||||||||||||||||||||||||||||||
| If there are more types of fractions, do like this also. | וכן אם היו מיני השברים יותר ככה תעשה | |||||||||||||||||||||||||||||||
|
דמיון [קבצנו][86] ב' שלישיות וג' רביעיות וד' חמישיות כמה יהיה המקובץ | |||||||||||||||||||||||||||||||
|
כפלנו ג' על ד' ונהיו י"ב עוד כפלנו הי"ב על הה' ונהיו ס' והוא המורה | |||||||||||||||||||||||||||||||
|
וב' שלישיותיו מ' | |||||||||||||||||||||||||||||||
|
וג' רביעיותיו מ"ה | |||||||||||||||||||||||||||||||
|
וד' חמישיותיו מ"ח | |||||||||||||||||||||||||||||||
|
והכל מקובצים קל"ג ערכנו אותם על ס' שהוא המורה והוא האחד השלם ונהיו שנים שלמים וחמישית אחת וחלק אחד מששים | |||||||||||||||||||||||||||||||
| Similarly, if you want to sum up more than these, proceed according to this rule. | וכן אם תרצה לקבץ יותר מאלה כן תעשה כמשפט הזה | |||||||||||||||||||||||||||||||
| Division of Fractions | ||||||||||||||||||||||||||||||||
| If you wish to divide fractions by fractions: | ואם תרצה לחלק נשברים על נשברים | |||||||||||||||||||||||||||||||
| Look for one denominator for both and take from it the number of parts required, then divide them by each other and the result is the sought. | בקש מורה אחד לשניהם וקח ממנו כמספר החלקים הדרושים וחלק זה על זה והעולה הוא המבוקש | |||||||||||||||||||||||||||||||
|
דמיון רצינו לחלק ד' תשיעיות על ה' שביעיות | |||||||||||||||||||||||||||||||
|
והמורה הוא ס"ג כי ט' פעמים ז' הם ס"ג | |||||||||||||||||||||||||||||||
|
וד' תשיעיותיו הם כ"ח | |||||||||||||||||||||||||||||||
|
וה' שביעיותיו הם מ"ה | |||||||||||||||||||||||||||||||
|
חלקנו הכ"ח על המ"ה ונהיו חצי אחד ותשיעית אחד וחלק א' מתשעים וזהו המבוקש | |||||||||||||||||||||||||||||||
| If there are integers and fractions, do as follows: | ואם היו שם שלמים עם שברים תעשה ככה | |||||||||||||||||||||||||||||||
| Seek for the denominator, which is the one unit, look at the [integer] and multiply it by it, add the fractions to it; do the same with the number, by which you divide; then divide by each other and the result is the required. | בקש המורה והוא הא' השלם ועליו תביט את מספר החלקים ותכפלם כמספרו ותחבר גם את השברים עמהם וכן תעשה גם במספר אשר תחלוק עליו אחר [87]כן חלק זה על זה והעולה הוא המבוקש | |||||||||||||||||||||||||||||||
|
דמיון רצינו לחלק ג' שלמים וב' חמישיות על ב' שלמים וד' שביעיות אחד | |||||||||||||||||||||||||||||||
|
והמורה ל"ה כי ה' פעמים ז' הם ל"ה והוא הא' השלם | |||||||||||||||||||||||||||||||
|
והג' שלמים הם ק"ה כי ג' פעמים ל"ה הם ק"ה והב' חמישיות הם י"ד כי חמישית הל"ה הם ז' חברנום עם הק"ה ונהיו קי"ט | |||||||||||||||||||||||||||||||
|
עוד שמנו הב' שלמים האחרים ע' והד' שביעיות כ' כי שביעית הל"ה הם ה' חברנום עם הע' ונהיו צ' | |||||||||||||||||||||||||||||||
|
חלקנו עליהם הקי"ט ונהיה אחד שלם ונשארו כ"ט חלקים שהם ב' תשיעיות מן הצ' ועשירית אחד | |||||||||||||||||||||||||||||||
| Subtraction of Fractions | ||||||||||||||||||||||||||||||||
| Similarly, if you wish to subtract fractions from fractions, do as follows: | וכן אם רצית לחסר שברים משברים ככה תעשה | |||||||||||||||||||||||||||||||
| First, seek for the denominator and see its parts, then subtract those from those. | בקש המורה בתחלה וראה חלקיו וחסר אלה מאלה | |||||||||||||||||||||||||||||||
|
דמיון רצינו לחסר ב' שביעיות מד' תשיעיות | |||||||||||||||||||||||||||||||
|
והמורה הוא ס"ג | |||||||||||||||||||||||||||||||
|
וב' שביעיותיו י"ח | |||||||||||||||||||||||||||||||
|
וארבע תשיעיותיו כ"ח | |||||||||||||||||||||||||||||||
|
תחסר י"ח מכ"ח ונשארו י' שהם פחות מששית מעט | |||||||||||||||||||||||||||||||
| Now, I will train you in the issue of the problems, so that you will be quick in your calculation. | ועתה ארגילך בענין השאלות כדי שתהיה זריז בחשבונך | |||||||||||||||||||||||||||||||
The Place of the Problems |
מקום השאלות | ||||||||
|---|---|---|---|---|---|---|---|---|---|
| Multiplication of Fractions | |||||||||
|
א כמה ט' חלקים מי"ג הכפולים על י"ז חלקים מי"ט | ||||||||
|
כי אלה אין להם ערך מדובר לכן ראוי שתדע איך תכפול ומה תעשה | ||||||||
|
ראוי שנדע בתחלה המורה והנה נכפול י"ג על י"ט יהיו רמ"ז והוא המורה | ||||||||
|
אחר כן נקח לכל א' מט' חלקים י"ט נהיו קע"א והוא המספר האחד | ||||||||
|
עוד נקח לכל א' מי"ז חלקים י"ג נהיו רכ"א | ||||||||
|
נכפול זה על זה נהיו ל"ז אלפים תשצ"א | ||||||||
|
חלקנו אותם על רמ"ז ועלו קנ"ג | ||||||||
|
והנה העולה מכפל ט' בי"ז גם כן קנ"ג | ||||||||
|
וערך זה אל רמ"ז כערך המספר הראשון אל מרובע רמ"ז וזהו מה שבקשנו | ||||||||
|
ואם תרצה לדקדקו חלק קנ"ג על י"ט יהיה העולה [ח'][88] חלקים מי"ג וישאר [שבר א' שהוא שבר חלק][89] מי"ט | ||||||||
|
או אם תרצה חלקנו הקנ"ג על י"ג ויהיו י"א חלקים שלמים מי"ט וי' שברים שי"ג מהם עושה חלק א' מי"ט | ||||||||
| How Much Problems | |||||||||
| Road | |||||||||
|
ב שאלה דרך חברנו אליה רביעיתה וחמישיתה והיתה י' מילין כמה הדרך | ||||||||
|
נשים המורה עשרים כי ד' פעמים ה' הם כ' | ||||||||
|
ורביעיתן וחמישיתן הן ט' נחברם עם המורה נהיו כ"ט | ||||||||
|
נכפול הכ' עם י' ונהיו ר' | ||||||||
|
נחלקם על כ"ט ונהיו ו' מילין וחצי מיל ושליש מיל וחלק א' [מט"ו][90] בקירוב וככה היא הדרך | ||||||||
| Money | |||||||||
|
ג שאלה לקחנו תשיעית ממון ועשיריתו וחלק א' מי"א ממנו כמה הוא כל זה מערך הממון | ||||||||
|
והנה זה נקל הוא מי שידע לחבר השברים כאשר כתבנו בחבורם | ||||||||
|
וכן נעשה נבקש מורה והוא שנכפול תשעה על עשרה ונהיו צ' עוד צ' על י"א ונהיו תתק"צ וזהו המורה | ||||||||
|
ותשיעיתו ק"י ועשריתו צ"ט וחלק אחד עשרה ממנו צ' והכל מחוברים רצ"ט | ||||||||
|
| |||||||||
|
וערך רצ"ט אל תתק"צ ככה ערך אלה אל הממון | ||||||||
|
חלקנו אותם אל צ' שהוא חלק אחד מי"א ונהיו ג' חלקים מי"א וכ"ט חלקים מצ' שהם שלישית חלק מי"א פחות משהו | ||||||||
|
ד שאלה ממון הוספנו עליו מחציתו שלישיתו חמישיתו וששיתו ובין הכל היו ששים כמה היה הממון | ||||||||
|
ידענו שהחצי והשלישית והששית הוא אחד שלם | ||||||||
|
ונחשב שהיה לו אחד ויש לו עתה שנים וחמשיתן | ||||||||
|
נחלק הששים על ב' והחמישית וכן היה הממון | ||||||||
|
והנה תשיב הב' [שלימי'][91] אל חמישיות מפני שיש לנו חמישית ונחבר גם החמישית שיש לנו עמם ונהיו י"א חמישיות | ||||||||
|
[נכפול][92] ה' על ס' ונהיו ש' והוא המורה | ||||||||
|
נחלקם על י"א והיה החלק כ"ז ושלשה חלקים מי"א וככה היה הממון | ||||||||
| How Many Problem - horses | |||||||||
|
ה שאלה אדם רוכב ראה איש מושך סוסים ואמר לו אנה אתה מוליך אלה הק' סוסים והשיב לו אלה ואחרים כמותם ומחציתם ורביעיתם ועם הסוס שאתה רוכב הם מאה | ||||||||
|
הנה נוציא הסוס שרוכב זה ונשארו צ"ט | ||||||||
|
יש לנו שנים ומחציתו ורביעית ומפני [93]שיש לנו רביעית נשיב הכל לרביעיות והנה הב' שלמים ח' רביעיות והמחצית ב' הרי י' נחבר גם הרביעיות שיש לנו עמם נהיו י"א רביעיות | ||||||||
|
אחר נשיב אותם אל הערכים ונכתוב אם הי"א רביעיות נתנו צ"ט הד' רביעיות שהוא האחד השלם כמה | ||||||||
|
נכפול האמצעיים שהם הד' על הצ"ט ונחלקם על י"א שהוא הקצה ונהיו ל"ו ככה מספר הסוסים | ||||||||
| Buy and Sell Problems | |||||||||
|
ו שאלה אדם קנה כ"ד ליטרא בכ"ד דינר ומכר הי"ב ליטרא ורביע ליטרא בדינר והי"ב ליטרין מכר ליטרא פחות רביע ליטרא בדינר נבקש לדעת אם הרויח או הפסיד | ||||||||
|
נשיב הי"ב ליטרא הראשונים לרביעיות ונהיו מ"ח | ||||||||
|
נחלקם על ה' כי ה' רביעיות מכר [בדינר][94] ונהיו ט' וחצי ועשירית ואלה הם הדינרים שלקח מהי"ב ליטרא הראשונים | ||||||||
|
עוד נכה הי"ב ליטרא [האחרוני'][95] בד' כי ליטרא פחות רביע הם ג' רביעיות מכר בדינר ונהיו מ"ח | ||||||||
|
לכן נחלקם על ג' ונהיו י"ו ואלה הם הדינרים שלקח מהי"ב ליטרא האחרונים | ||||||||
|
נחבר שני [הממונות][96] נהיו כ"ה וחצי ועשירית | ||||||||
|
נוציא כ"ד דינר של הקרן נשאר דינר וחצי ועשירית וזהו הריוח | ||||||||
|
| |||||||||
|
ז שאלה אדם קנה ג' חמישיות ליטרא בפשוט ומכר ד' שביעיות ליטרא בפשוט והרויח פשוט כמה היה ממונו | ||||||||
|
נבקש המורה והוא ל"ה | ||||||||
|
וג' חמישיותיו כ"א | ||||||||
|
וד' שביעיותיו כ' | ||||||||
|
והממון היה כ' פשוטים כי בכל פשוט ירויח חלק אחד מכ' בפשוט וכשימכור של כ' פשוטים ירויח פשוט אחד | ||||||||
|
שאלה אדם קנה ט' חלקים מי"ז חלקי ליטרא בפשוט ומכר י' חלקים מי"ט בפשו' והרויח פשוט כמה היה הממון | ||||||||
|
נבקש המורה והוא שנכפול י"ז על י"ט ונהיו שכ"ג | ||||||||
|
כי ידוע כי ט' חלקים מי"ז הם קע"א וככה היה הממון | ||||||||
|
[וי'][97] חלקים מי"ט הם ק"ע | ||||||||
|
והנה הרויח פשוט | ||||||||
| How Much Problem - Money | |||||||||
|
ח שאלה ממון חברנו שלישיתו רביעיתו חמישיתו והיו שלשים כמה הוא כל הממון | ||||||||
|
נבקש המורה והוא ששים | ||||||||
|
ושלישיתו כ' ורביעיתו ט"ו וחמישיתו י"ב וכלם מ"ז | ||||||||
|
הנה כערך מ"ז אל ששים כן ערך שלשים אל כל הממון | ||||||||
|
ונכתוב ככה אם המ"ז יתנו ס' השלשים מה יתנו | ||||||||
|
| ||||||||
|
נכפול האמצעיים ונהיו אלף ת"ת | ||||||||
|
נחלקם על הקצה שהוא המ"ז נהיה החלק ל"ח ורביעית וב' חלקי' ורביע חלק ממ"ז | ||||||||
| Partnership Problem - For the Same Time | |||||||||
|
ט שאלה אם אנשים היו חמשה נתן הא' ו' דינרי' והשני ז' דינרים והשלישי ח' דינרים והרביעי ט' דינרים והחמישי י' דינרים והרויחו בין הכל י"א דינרים | ||||||||
|
הנה אלה הי"א דינרי' הרויחו אותם כל הדינרים מקובצים | ||||||||
|
ונראה אותם כמה הם אחר כן נשיב אותם אל הערך של כל אחד ואחד וכפי ערך ממונו ככה יקח | ||||||||
|
והנה הדינרים מקובצים הם מ' דינר | ||||||||
|
ונכתוב הערך של הראשון ככה | ||||||||
|
| ||||||||
|
אם המ' דינר הרויחו י"א הו' כמה ירויחו | ||||||||
|
נכפול האמצעיים ונהיו ס"ו | ||||||||
|
ונחלקם על מ' שהוא הקצה ונהיה החלק הא' אחד וחצי ושמיני' וחלק אחד מארבעים וככה יקח בעל הו' דינרים | ||||||||
|
עוד נכתוב הערך של השני ככה | ||||||||
|
| ||||||||
|
אם המ' דינר הרויחו י"א הז' דינרים כמה ירויחו | ||||||||
|
נכפל האמצעיים ונהיו ע"ז | ||||||||
|
נחלקם על הקצה שהוא המ' ונהיה החלק הא' אחד וג' רביעיות ושמינית וחלק אחד מעשרים וככה יקח בעל הז' דינרים | ||||||||
|
עוד נכתוב הערך של השלישי ככה | ||||||||
|
| ||||||||
|
אם המ' דינרי' הרויחו י"א הח' דינרים כמה ירויחו | ||||||||
|
נכפל האמצעיים ונהיו פ"ח | ||||||||
|
נחלקם על המ' שהוא הקצה ונהיה החלק האחד ב' וחמישית וככה יקח בעל הח' דינר | ||||||||
|
עוד נכתוב ערך הרביעי כך | ||||||||
|
| ||||||||
|
[98]אם המ' דינר הרויחו י"א הט' כמה ירויחו | ||||||||
|
נכפול האמצעיים ונהיו צ"ט | ||||||||
|
נחלקם על המ' שהוא הקצה ונהיה החלק הא' ב' ורביעית וחמישית וחלק א' מארבעי' וככה יקח בעל הט' דינר | ||||||||
|
עוד נכתוב הערך של החמישי ככה | ||||||||
|
| ||||||||
|
אם המ' דינרים הרויחו י"א הי' כמה ירויחו | ||||||||
|
נכפול האמצעיים ונהיו ק"י | ||||||||
|
נחלקם על מ' שהוא הקצה ונהיה החלק האחד ב' ושלש רביעיות וככה יקח בעל הי' דינרים | ||||||||
|
וכשתקבץ כל החלקים שיקחו הכל תמצאם י"א לא פחות ולא יתר | ||||||||
|
| |||||||||
|
דרך אחרת למצוא זה | ||||||||
|
תעשה הי"א דינר שהם הריוח כלם פשוטים ותחלקם על מ' ותראה כמה ריוח יגיע לכל דינר מהמ' | ||||||||
|
והריוח ההוא תכפול אותו עם הו' ואשר יהיה ככה יגיע לבעל הו' | ||||||||
|
עוד תכפול אותו עם הז' ואשר יהיה ככה יגיע לבעל הז' | ||||||||
|
וכן תעשה עם הח' והט' והי' | ||||||||
| Purchase Problem - Equal Amount | |||||||||
|
י שאלה יש אצל הקונה ג' מטבעים והלך אצל המוכר [לקנות][99] ליטרא אחת משי | ||||||||
|
נבקש המורה כי הוא ע"ב שהרי כפל ג' על ד' י"ב וכפל י"ב על ו' ע"ב | ||||||||
|
ושלישיתו כ"ד ורביעיתו י"ח וששיתו י"ב והכל חמשים וארבע וזהו הדינר | ||||||||
|
נחלק המורה שהוא ע"ב על חמשים וארבע ונהיה החלק הא' א' [ושליש][100] וככה יקח מכל מטבע | ||||||||
|
והנה נעשה הדינר י"ב פרוטות ויקח י"ו פרוטות מכל מטבע | ||||||||
|
ואם תרצה לדעת איך הוא כך | ||||||||
|
תחשוב כאלו לקח ל"ו [פרוטות][101] ממטבע ששוה ג' דינרים ולקח ממנו י"ו פרוטו' | ||||||||
|
וכשלקח עוד י"ו פרוטות ממטבע ששוה ד' דינרים כאלו לקח י"ב פרוטות ממטבע ששוה ג' דינרים כערך הד' אל הג' | ||||||||
|
נחבר הי"ב עם הי"ו נהיו כ"ח | ||||||||
|
עוד כשלקח י"ו פרוטות ממטבע ששוה ו' דינרים הוא כאלו לקח ח' פרוטות ממטבע ששוה ג' דינרים כערך הג' אל הו' | ||||||||
|
נחבר הח' עם הכ"ח ונהיו ל"ו וזהו המבוקש | ||||||||
| Payment Problems | |||||||||
| constructing a shelf | |||||||||
|
יא שאלה אדם התנה עם אומן לבנות לו אצטבא ו' באורך ו' ברחב ויתן לו ח' דינרים והוא בנה לו ג' באורך ג' ברחב | ||||||||
|
אין ספק כי יתן לו הרביעי' שהוא ב' דינרים כי כפל חצי [על חצי][102] הוא רביעית אחד | ||||||||
|
שאלו היה בונה כל האורך עם חצי הרחב או ההפך היה נותן לו המחצית | ||||||||
|
אבל אם התנה עמו שיבנה לו ה' באורך ו' ברחב ז' בגובה ויתן לו ח' דינרים והוא בנה ד' באורך [וה' ברחב][103] ו' בגובה | ||||||||
|
אז ראוי שנחבר כל מספרי התנאי ונשיבם אל הערכים וכן מספרי הבנין | ||||||||
|
חברנו בתחלה מספרי התנאי ונהיו י"ח | ||||||||
|
עוד חברנו מספרי הבנין ונהיו ט"ו | ||||||||
|
ועשינו הערך ככה אם הי"ח יתנו ח' דינרי' הט"ו כמה יתנו | ||||||||
|
| ||||||||
|
כפלנו האמצעיים ונהיו ק"כ | ||||||||
|
חלקנום על הי"ח שהוא הקצה ונהיה החלק ו' וב' שלישיות וככה יקח | ||||||||
| three workers, three different daily wages, the same actual payment | |||||||||
|
יב שאלה אדם א' שכר שלשה אחים ראובן שמעון לוי לעבוד אחד מהם עמו ב' ימים ואם יעבוד ראובן עמו כל הב' ימים יתן לו ו' דינרי' | ||||||||
|
נבקש המורה והוא ע"ב כי כפל ו' על ד' הם כ"ד ואלה על ג' הם ע"ב | ||||||||
|
וששיתו ורביעיתו ושלישיתו הם נ"ד | ||||||||
|
[הנה כערך ע"ב אל נ"ד][104] ככה יקח [105]מהדינרים וערך ע"ב אל נ"ד הוא אחד ושליש וככה יקח כל אחד ואחד | ||||||||
|
נבקש לדעת כמה שעות עבד כל [אחד ואחד][106] | ||||||||
|
ונשיבהו אל הערכים ונשיב הדינר שלישיות מפני השליש שעודף על הדינרים | ||||||||
|
והיום י"ב שעות | ||||||||
|
ונעשה ערך ראובן כך אם הי"ח שלישיות כ"ד שעות הד' שלישיות כמה יתנו | ||||||||
|
ואז נכפול האמצעיים ונהיו צ"ו | ||||||||
|
נחלקם על י"ח ונהיה החלק ה' שעות ושליש וככה עבד ראובן | ||||||||
|
| ||||||||
|
ונעשה ערך שמעון כך אם הי"ב שלישיות כ"ד שעות הד' שלישיות כמה יתנו | ||||||||
|
| ||||||||
|
כפלנו האמצעיים והם צ"ו | ||||||||
|
חלקנום על י"ב ונהיה החלק ח' שעות וככה עבד שמעון | ||||||||
|
ונעשה ערך לוי כך אם הט' שלישיות יתנו כ"ד שעות הד' שלישיות כמה יתנו | ||||||||
|
| ||||||||
|
כפלנו האמצעים ונהיו צ"ו | ||||||||
|
חלקנום על ט' ונהיה החלק י' שעות וב' שלישיים וככה עבד לוי | ||||||||
|
חבר כל השעות שעבדו שלשתם ותמצאם כ"ד שעות שהם שני ימים | ||||||||
| Boiling Problem | |||||||||
|
יג שאלה אדם אחד היה מבשל משקה ו' מדות ורצה שישאר השליש ונחסרה המדה האחת בבשול אחר נשפכה מדה אחת ונשארו הד' מדות ורוצה להשאירם כמשפט הראשון | ||||||||
|
נשיבם אל הערכים ככה אם הה' יתנו ב' הד' כמה יתנו
| ||||||||
|
נכפול האמצעיים ונהיו ח' נחלקם על הקצה שהוא הה' ונהיה החלק א' וחצי ועשירית וככה ראוי שישאר | ||||||||
|
יד שאלה יריעה מאה אמות והתחיל למנותה ראובן מן הקצה האחד ושמעון מן הקצה הא' | ||||||||
|
נחבר שני המנינים אשר הם כ"ב אמות בשעה ונחלק הק' אמות עליהם ונהיה החלק ד' וחצי וחלק א' מכ"ב וידענו שבד' שעות וחצי וחלק מן כ"ב בשעה יפגשו שניהם על המנין | ||||||||
|
ואם תרצה לדעת כמה אמות מנה ראובן | ||||||||
|
תכפול י' בד' וחצי וחלק אחד מכ"ב ואשר יעלה כן מספר האמות אשר מנה ראובן | ||||||||
|
וכן אם תרצה לדעת כמה מנה שמעון | ||||||||
|
תכפול הד' שעות וחצי וחלק מכ"ב על י"ב ואשר יעלה כך מספר האמות אשר מנה שמעון | ||||||||
| Shared Work Problem - Draining a Vessel - Barrel | |||||||||
|
טו שאלה חבית מלאה יש בה שלשה נקבים אם תפתח האחד יכלה החבית בחצי היום | ||||||||
|
אתה צריך לדעת כי היום י"ב שעות | ||||||||
|
וכן החבית כמה מדות מכילה ונניח שמכילה מ"ח מדות | ||||||||
|
והנה בפתיחת הנקב הא' יעלה בו' שעות שהם ח' מדות בשעה | ||||||||
|
ובפתיחת הנקב השני יכלה בד' שעות שהם י"ב מדות בשעה | ||||||||
|
ובפתיחת הנקב השלישי יכלה בג' שעות שהם י"ו מדות בשעה | ||||||||
|
תחבר כל המדות אשר יכלו בשעה אחת והם ל"ו | ||||||||
|
תחלק המ"ח על הל"ו יהיה אחד ורביע [נ"א ושליש][107] והנה בשעה א' ורביע [ושליש][108] יכלה הכל | ||||||||
|
ונעשה הערכים ככה אם הל"ו שעה אחת המ"ח על כמה יהיה | ||||||||
|
| ||||||||
|
נכפול האמצעיים והם מ"ח נחלקם על הקצה שהם ל"ו ונהיה החלק הא' אחד ושלישית | ||||||||
| Find a Quantity Problems - Whole from Parts Problems | |||||||||
|
טז שאלה רומח חציו במים שלישיתו בעפר ולמעלה ו' אמות כמה גבהות כל הרומח | ||||||||
|
נבקש המורה והוא ו' | ||||||||
|
וחציו ושלישיתו ה' | ||||||||
|
חסרם מן הו' ונשארו א' | ||||||||
|
נכפול הו' עם הו' שהם האמצעיים | ||||||||
|
ונחלקם על הא' שהוא הקצה נהיו ל"ו וככה גובה הרומח | ||||||||
|
וחציו י"ח ושלישיתו י"ב הרי ל' ולמעלה ו' | ||||||||
|
ונעשה הערך כך אם הא' יתנו ו' הו' כמה יתנו | ||||||||
| |||||||||
|
יז שאלה אילן חמישיתו בעפר וששיתו במים ולמעלה מהמים ז' אמות כמה גבהות כל האילן | ||||||||
|
[109]הנה המורה ל' | ||||||||
|
וחמישיתו וששיתו י"א | ||||||||
|
נגרעם מל' שהוא המורה נשארו י"ט | ||||||||
|
נכפול האמצעיים שהם הז' בל' נהיו ר"י | ||||||||
|
נחלקם על י"ט ונהיו י"א חלקים וחלק א' מי"ט וככה גבהות האילן | ||||||||
|
ונעשה ערך כך | ||||||||
|
| ||||||||
| Triangulation Problem - Two towers | |||||||||
|
יח שאלה אם היו שני מגדלים גובה האחד ששים אמות וגובה השני מ' אמות והמרחק שביניהם נ' אמה | ||||||||
|
נחבר מרובע מ' שהוא אלף ת"ר עם מרובע נ' שהוא אלפים ות"ק נהיו ד' אלפים ק' אחר כן נקח מרובע ס' שהוא ג' אלפים ות"ר ונגרעם מן שני המרובעים הנזכרים שהם ד' אלפים ק' ונשארו ת"ק והוא המורה | ||||||||
|
| |||||||||
|
נכפול גם מרחק שני המגדלי' שהוא נ' על ב' ונהיו ק' נחלק המורה עליהם ונהיו ה' וככה מרחק הבריכה מן המגדל שגבהו ס' אמות | ||||||||
|
ומן המגדל האחר המ"ה אמות הנשארות | ||||||||
| Joint Purchase Problem - If You Give Me - Buying a Fish | |||||||||
|
[יט] שאלה שלשה אנשים אשר הלכו בשוק לקנות דג אמר הא' אני אתן כל מה שיש לי ואתם תנו החצי ממה שיש לכם ונקח הדג | ||||||||
|
כתוב דבר הקונים החצי והשליש והרביע כזה
| ||||||||
|
אחר כן תאמר מהו כאשר נקח חציו ממנו ישאר ג' כמספר הקונים והנה הוא הו' כתוב אותו תחת הב' | ||||||||
|
עוד תאמר מהו כאשר תקח שלישיתו ממנו ישאר ג' כמספר הקונים והנה הוא ד' וחצי כתוב אותו תחת הג' | ||||||||
|
עוד תאמר מהו כאשר תקח רביעיתו ממנו ישאר ג' כמספר הקונים והנה הוא ד' תכתבהו תחת הד' | ||||||||
| |||||||||
|
כפול הכל עם הב' ותאמר ב' פעמים ו' הם י"ב עוד ב' פעמים ד' וחצי הם ט' | ||||||||
|
כפול הראשון שהוא י"ב עם ב' וחסרהו מן המורה נשארו ה' וכך יש לראשון בכיסו | ||||||||
|
כפול השני שהוא ט' עם הב' וחסרהו מן המורה נשארו י"א וכך יש לשני בכיסו | ||||||||
|
כפול השלישי שהוא ח' עם ב' וחסרהו מן המורה ונשארו י"ג וכך יש לשלישי בכיסו | ||||||||
|
נמצא שקנו הדג בי"ז | ||||||||
|
ואם תרצה לבחון זה | ||||||||
|
תביט כי הראשון נתן כל מה שיש לו והיו ה' והם נתנו החצי שיש להם ומה שיש להם הם כ"ד כי השני יש לו י"א והג' י"ג וחציים הוא י"ב תחברם עם הה' ונהיו י"ז [והוא מחיר הדג][111] | ||||||||
|
| |||||||||
|
עוד השני נתן כל אשר לו והם י"א והראשון והשלישי נתנו השליש ממה שיש להם שהם ו' כי לשניהם י"ח לראשון ה' ולשלישי י"ג חבר הי"א עם הו' ונהיו י"ז והם מחיר הדג | ||||||||
|
| |||||||||
|
עוד השלישי נתן כל אשר לו והם הי"ג והראשון עם השני נתנו ד' שהוא הרביע ממה שיש להם כי לראשון ה' ולשני י"א ומחיר הדג י"ז | ||||||||
|
| |||||||||
| Divide a Quantity Problem - Proportional Division – Promissory Note | |||||||||
|
[כ] שאלה אדם אחד הוציאו עליו שטר חוב ארבעה אנשים הראשון אם יעבוד עמו חדש ימים יתן לו עשרה זהובים | ||||||||
|
הנה חכמי החשבון יתנו לכל אחד כפי ערך ממונו | ||||||||
|
והנה המורה עשרים וארבעה כי תכפול הב' על השלשה והם ו' עוד הו' על הד' הם כ"ד ובזה ימצאו כל החלקים הנזכרים ונשים האחד השלם כ"ד כי הוא המורה | ||||||||
|
וכשתחבר אליו חציו ושלישיתו ורביעיתו יהיה בין הכל נ' | ||||||||
|
ונעשה הי' זהובים ק"כ פשוטים מי"ב פשוטים בזהוב | ||||||||
|
ונעשה ערך הראשון [112]כך אם הנ' יתנו לו כ"ד הק"כ מה יתנו לנו | ||||||||
|
| ||||||||
|
נכפול האמצעיים ונהיו ב' אלפים תת"פ | ||||||||
|
נחלקם על הנ' שהוא הקצה נהיה החלק נ"ז פשוטים וחצי פשוט ועשירי' פשוט וזהו החלק הראשון בעל הי' זהובים | ||||||||
|
ונעשה הערך השני כך אם הנ' יתנו לנו י"ב הק"כ מה יתנו | ||||||||
|
| ||||||||
|
נכפול האמצעיים ונהיו אלף ת"מ | ||||||||
|
נחלקם על הנ' שהוא הקצה ועלו כ"ח פשוטי' וחצי פשוט וחמשית פשוט ועשירית פשוט וזהו חלק השני בעל הה' זהובים | ||||||||
|
ונעשה ערך השלישי ככה אם הנ' יתנו ח' הק"כ כמה יתנו | ||||||||
|
| ||||||||
|
נכפול האמצעיים ונהיו תתק"ס | ||||||||
|
נחלקם על הנ' שהוא הקצה יהיו י"ט פשוטים וחמישית וזהו החלק השלישי בעל הג' זהובים ושליש | ||||||||
|
ונעשה ערך הרביעי ככה אם הנ' יתנו ו' הק"כ כמה יתנו | ||||||||
|
| ||||||||
|
נכפול האמצעיים ונהיו תש"כ | ||||||||
|
נחלקם על הנ' שהוא הקצה ונהיו י"ד פשוטים וב' חמישיות פשוט וזהו החלק הרביעי בעל הב' זהובים וחצי | ||||||||
|
וכשתחבר כל כל אלה החלקים יעלו ק"כ פשוטים שהם י' זהובים | ||||||||
|
| |||||||||
|
ואם לא תרצה להשיב הכל אל הערכים מפני הטורח תעשה כך | ||||||||
|
אחר שידעת חלק הא' מן הערכים תן מחציתו לב' ושלישיתו לג' ורביעיתו לד' ושוה יהיה זה וזה | ||||||||
| You can create other questions endlessly and you can answer any of them, if you know the rules we elaborated in this book. | וכן תוכל אתה לעשות שאלות אחרות עד אין קץ ותוכל להשיב על כל אחת מהן אם תדע הכללים שהקפנו בהם בזה החבור | ||||||||
| I have not mention a way to find the roots of the squares here, because they are not needed in this book, so I have postponed them to their place, where I will explain them, if the One Who gives strength to the weary [Isaiah 40, 29] wants. | ולא הזכרתי דרך מציאת שרשי המרובעים הנה כי אינם צורך בזה החבור ואחרתים עד מקומם ושם אבארם אם יחפוץ הנותן ליעף כח[note 2][113] | ||||||||
The Second Book of this Treatise Discusses Geometry |
[114]הספר השני מזה החבור המדבר בחכמת המדות | |
[The First Section] |
||
Chapter One of the First Section of the Second Book |
הפרק הראשון מהחלק הראשון מהספר השני | |
| Mordechai said: before discussing geometry [lit. the science of measurement] it is appropriate to explain the names used by the masters of this craft and science, for in each science, the masters of this science use specific names that indicate issues of this science, not issues that are indicated in other than this science, as they are conventional. | אמר מרדכי קודם שנדבר על חכמת המדות ראוי לבאר שמות שנשתמשו בהם בעלי זאת המלאכה והחכמה כי לכל חכמה שמות מיוחדות נשתמשו בהם בעלי החכמה ההיא מורות בענינים באותה החכמה בלתי הענינים אשר יורו בזולת החכמה ההיא להיות שהם הסכמיים | |
| As they are used in other than that science, they can be transferred to a specific science, either by metaphor or by analogy or by the translation, and sometimes even by complete similarity. | וכאשר הניחו בזולת החכמה ההיא על מה שהניחו כן יוכלו להעתיקם ממה שהניחום למה שהניחום בחכמה מיוחדת אם על דרך השאלה או על דרך הספוק או על דרך ההעתקה ולפעמים גם ע"ד השתוף הגמור | |
| Therefore, if an author of a book wants his words to be understood, he should first explain the words used by the masters of this science. | ולכן ראוי למחבר ספר אם ירצה להיות דבריו מובנים לבאר תחילה השמות אשר נשתמשו בם בעלי החכמה ההיא | |
| So did the sage Euclid in the Book of Elements, who clarified in the introduction of each book the things that are necessary in that book. | וכן עשה גם החכם אקלידס בספר היסודות אשר ביאר בהצעת כל מאמר דברים הצריכים באותו מאמר | |
|
ונאמר שכשתשמע הנה נקדה תבין שתורה על דבר שאין לו שעור כלל לא ארך ולא רחב ולא עומק והיא נמצאת בראש הקו כי היא תכליתו וכן היא אשר תמצא באמצע הקו כשתשער רמיזה היא תכלית חצי הקו והיא בעצמה ראש חצי הקו האחר | |
|
והקו הוא אורך בלא רחב נמשך בין שתי נקדות אשר הן תכליותיו | |
|
והשטח הוא הרחב המתוח בין הקוים ויש לו אורך ורחב לבד ותכליותיו הם הקוים | |
|
והזוית השטוחה היא אשר יקיפו בה שני קוי' ישרי' | |
|
והזוית ממנה נצבת וממה חדה וממנה נרוחת | |
|
והזוית הנצבת היא כשיפול הקו הישר על קו ישר וישים השתי זויות אשר משתי צדדיו שוות כזה ┴ | |
|
והחדה היא אשר היא יותר קטנה מזאת | |
|
והנרוחת היא אשר היא יותר גדולה מזאת | |
|
והקו אשר יפול על הקו ויעשה שתי הזויות אשר משתי צדדיו שוות יקרא עמוד | |
|
והקו שהוא עומד עליו יקרא תושבת | |
|
והקוים הנכחיים הם אשר כשיוצאו לכל א' משני צדדים ואפילו ללא תכלית לא יפגשו | |
|
והתמונה השטחית תחלק אל ישרת הקוים ואל בלתי ישרת הקוים | |
|
והתמונה הישרת קוים היא אשר יקיפו בה ג' קוים או יותר | |
|
ואשר יקיפו בה ג' קוים לא יותר תקרא משולש | |
|
ואשר יקיפו בה ד' קוים תקרא מרובע | |
|
ואשר יקיפו בה ה' קוים תקרא מחומש | |
|
וכן כל כיוצא בזה | |
|
והקוים אשר יקיפו בתמונה יקראו צלעות | |
| The triangles are divided into three species: | והמשולש יחלק אל שלשה מינים | |
|
הא' יקרא שוה הצלעות והוא אשר שלש צלעותיו שוים | |
|
והשני יקרא שוה השוקים והוא אשר תהיינה שתי צלעותיו שוות והשלישית בלתי שוה אם יותר ארוכה מכל אחת מהן או יותר קצרה מכל אחת מהן | |
|
והשלישי יקרא מתחלף הצלעות והוא אשר אין אחת שוה לחברתה | |
The triangles are also divided into:
|
עוד [115]המשולש יחלק אל נצב הזויות והוא אשר זויתו האחת נצבת כזה | |
|
ואל נרוחת הזוית והוא שזויתו האחת מרווחת כזה | |
|
ואל חד הזויות והוא אשר שלש זויותיו חדות | |
| The quadrangles are divided into: equilateral quadrangle and non-equilateral quadrangle. | ובעל הד' צלעות יחלק אל מרובע שוה הצלעות ואל בלתי שוה הצלעות | |
|
עוד השוה הצלעות יחלק אל מה שהוא נצב הזויות ויקרא שוה הצלעות נצב הזויות כזה | |
|
ואל מה שהוא בלתי נצב הזויות כזה ויקרא מעויין | |
|
עוד הבלתי שוה הצלעות יחלק אל מה שהוא שתי צלעותיו הנכוחיים שוים וזויותיו נצבות ויקרא מרובע ארוך כזה | |
|
ואל [מה שכל שתי][116] צלעותיו [הנכוחות][117] שוות אבל אין זויותיו נצבות ויקרא דומה למעויין כזה | |
|
ואל זולת אלה ויקרא הנוטה | |
|
והוא ארבע תמונות יתבארו בזה החבור | |
|
והתמונה הבלתי ישרת הקוים השטוחה תחלק אל עגולה וחצי עגולה | |
|
והעגולה היא תמונה יקיף בה קו אחד בתוכה נקדה כל הקוים היוצאים ממנה אל המקיף שוים | |
|
והנקדה ההיא תקרא מרכז העגולה | |
|
והקו [הישר][118] אשר יצא ממקיף העגולה ויעבור על המרכז [ויצא עד המקיף מהצד האחר יקרא הקוטר | |
|
ואם לא יעבר על המרכז][119] יקרא מיתר | |
|
והחתיכה אשר תושבתה במיתר יקרא קשת | |
|
וחצי העגולה היא אשר יקיף בה חצי הקו המקיף והאלכסון | |
|
וחתיכת העגולה היא תמונה תקיף בה היתר והקשת ותחלק לשני מינים אם שהיא קטנה מחצי עגולה או גדולה הימנה | |
|
ותשבורת התמונה הוא החלקים המרובעים נצבי הזויות אשר ארכם כרחבם או השוים להם הממלאים את התמונה הדרושים על התמונה | |
|
וההכאה היא כפל צלע בצלע או קו ישר בקו קשתי או מספר במספר | |
| Euclid has already explained in a book that he wrote about the measurement of land that the plane geometry is based on four things: directions, points, lines, and angles; and it also has species and types. | וכבר ביאר אקלידס בספר אחד שחבר במדידת הארץ כי חכמת המדות השטוחה היא מיוסדת מארבעה דברים מהפאות ומהנקודות ומהקוים ומהזויות וגם כן היא מקבלת סוגים ומינים | |
|
והפאות הם מזרח ומערב וצפון ודרום | |
|
והנקדות הם אשר תקח אותם התחלה או סימן לדבר | |
|
והקוים הם י' קו ישר ותושבת וראש ועמוד וקו נכחי וקטר ושוקים ומקיף ואלכסון ומיתר | |
|
והקו הישר הוא הנמשך על יושר משתי תכליותיו שהם שתי נקדות | |
|
והתושבת היא קו ישר אחד עומד עליו קו ישר אחר ועושה השתי זויות אשר משני צדדיו נצבות | |
|
והראש הוא הקו העומד על התושבת | |
|
והעמוד הוא הקו היורד מהראש אל התושבת ועושה השתי זויות אשר משתי צדדיו נצבות | |
|
והקו הנכחי הוא קו עומד נכח קו אחר ומרחקי קצותיהם אשר על זויות נצבות שוים | |
|
והקטר הוא הקו הנמשך מזויות המרובעים וכיוצא בהם עם הזוית האחרת | |
|
והשוקים הם קוים ישרים יורדים מקצות [הראש][120] עד שתי קצות התושבת | |
|
והמקיף הוא הקו הסובב במרחק שהוא [מהמרכז][121] עד הקו ההוא והקוים היוצאים אליו מהמרכז שוים | |
|
והאלכסון הוא [122]קו יוצא מהמקיף ועובר על מרכזו ויוצא אל המקיף מהצד האחר וחולק את המקיף בשני חלקים שוים | |
|
והמיתר הוא הקו הישר אשר תחת הזוית הנצבת | |
|
והזויות הם ג' נצבת חדה ונרוחת | |
|
הנצבת היא כאשר יעמוד קו ישר על קו ישר ויעשה הזויות אשר משני צדדיו שוות כל אחת תקרא נצבת | |
|
והזוית אשר היא קטנה מזאת תקרא חדה | |
|
ואשר היא גדולה מזאת תקרא נרוחת | |
|
סוגי המדידה הם שלש [מדידות הקוים][123] הישרי' ומדידת התמונה ר"ל תשבורת התמונות ומדידת הגופנים | |
|
מדידת הקוים הישרים היא [המדידה][124] אשר ימדדו על יושר באורך לבד וזה יקרא מדידה מספרית | |
|
מדידת תשבורת התמונות [הוא אשר יש לו אורך ורחב כי ממנו יודעו תשברת התמונות ותקרא גם כן מדידה כחנית | |
|
מדידת הגופניים] הוא אשר יש לו אורך ורחב ועובי כי ממנו יודע מדידת הגופנים ויקרא גם כן מעוקב | |
|
ומיני המדידה הם חמשה המרובע והמשולש והמעוין והנוטה והעגולות | |
|
והתמונות י"ח | |
|
אם תמונות המרובעים ב' מרובע נכחי הצלעות ונצב הזויות | |
|
ואם תמונות המשולשים [ששה][125] משולש שוה הצלעות ומשולש שוה השוקים ומשולש מתחלף הצלעות ומשולש נצב הזויות ומשולש נרוח הזויות ומשולש חד הזויות | |
|
ואם תמונות המעויינים ב' המעויין והדומה למעויין | |
|
ואם תמונות הנוטים הם ד' נוטה נצב הזויות נוטה שוה השוקים נוטה חד הזויות נוטה נרוח הזויות | |
|
ואם תמונות העגולות ד' העגולה וחצי העגולה וחתיכת העגולה [הגדולה][126] מחציה ומעלה וחתיכת העגולה הקטנה שהיא קטנה ממנה | |
Chapter Two [on the Measuring of the Various Quadrangles] |
הפרק השני | |
| Know that the triangle is the basis and foundation for all the planar shapes that consist of straight lines, for they are composed of it and decomposed to it, as Nicomachus of Gerasa stated in the second section of the Introduction to Arithmetic he wrote. | דע כי המשולש הוא שרש ופנה לכל התמונות השטחיות ישרות הקוים כי ממנו חוברו ואליו יותכו כאשר הודיע זה ניקומאכוש הגיהרשיני במאמר השני מספר הארתימישיקא שחבר | |
| Therefore, whoever knows how to measure the triangles knows how to measure all the shapes by multiplying the area of the triangle by the number that generates the change if they are regular and if they are irregular it is by summing the areas of all the triangles that generate them. | ולכן מי שידע למדוד המשולשים ידע למדוד כל התמונות בכפל תשבורת המשולש במספר אשר יולידו התמורה אם הם שוים ואם היו בלתי שוים יהיה זה בקבוץ תשבורת כל המשולשי' אשר יולידוהו | |
| Because of this it is enough to present the measuring of the triangle alone, however because the measure and the size by which we measure is a square, the length of which is equal to its width, for the area of the shapes is measured by the square cubits, since we know its definition, and the size of the triangle is verified only in comparison with the square, because its demonstration is obtained by comparison with the square. Hence, we should first introduce the measuring of the [squares], then we shall state the methods of the triangles and the others. | ומפני זה היה מספיק להודיע מדידת המשולש למנין לבד אבל מפני שהמדה והשעור אשר נשער בה מרובעת ארכה כרחבה כי תשבורת התמונות הם משוערות על האמות המרובעות כאשר הודענו מן גדרה והמשולש בעצמו לא יתאמת מנינו רק בהצטרפו אל המרובע כי המופת שיובא עליו בהקשה אל המרובע לכן ראוי שנודיע מדידת המשולשים [נ' המרובעים] תחלה אח"כ נודיע דרכי המשולשים והשאר | |
| Before that, we announce that every unknown is obtained only through the known, therefore all those that are required are raised to the axioms or to one of the species which are known by themselves. | וקודם זה נודיע שכל מוסכל לא יגיע אלא בידוע ולכן עלו כל הדרושים אל המושכלות הראשונות או אל אחד מהמינים אשר הם ידועים בעצמם | |
| It is known that when we seek to find the area of any shape, it is unknown to us before we find it. | וידוע כי כשנדרוש למצוא תשבורת תמונה איזו שתהיה קודם שנמצאנה היא מוסכלת אצלנו | |
| Therefore, we should obtain it through the known and the known that we obtain is the knowledge of a part of it, whether one of its sides, its height, its base, or other than these. | ולכן ראוי להגיעה לנו בידוע והידוע אשר יגיע לנו היא ידיעת חלק ממנה אם צלעות מצלעותיה או עמוד ותושבת וזולת זה | |
| If these are also unknown, then we obtain the cubits by which they are measured, so we should say that the known cubit, by which we obtain the measured that are unknown, is only length, without extension, it is not a square cubit, as the cubits of the area, for the ends of the square [cubit] are also lines that are only length and they limit it. | ואם גם אלה הם מוסכלים אז תגיע לנו האמה אשר ימדדו בה ולכן ראוי להודיע [127]שהאמה הזאת ידועה אשר תגיע לנו ידיעת המוסכלים מהנמדדים היא אורך לבד בלי מרחב ואינה אמה מרובעת כאשר הם אמות התשבורת כי גם המרובעת תכליותיה הם הקוים אשר הם אורך לבד והם המגבילים אותה | |
| From here I begin to explain how the quadrangles are measured and we start from the right-angled equilateral square, because it is as a foundation for the rest of the quadrangles, since the rhombus is similar to it by its equal sides and the rectangle by its right angles. | ומהנה אתחיל לבאר איך ימדדו המרובעים ונתחיל ממרובע שוה הצלעות נצב הזויות כי הוא כשרש לשאר המרובעים אחרי שהמעויין ידמה לו בשווי הצלעות והארוך בהתיצבות הזויות | |
| The cubit of the area is square, equilateral and equiangular. | ועוד כי אמת התשבורת היא מרובעת הצלעות שוים והזויות | |
| It is a cubit of the area, because it demonstrates for us the measure of the shape and the thing that demonstrates the measure of the thing should be equally known. | והיתה זאת אמת התשבורת כי זאת תודיע לנו השעור של התמונה והדבר אשר יודיע שעור הדבר ראוי שיהיה שוה נודע | |
| It is known that the right angles are always equal, which is not the case for acute angle and obtuse angle that can be smaller or greater; and the thing that can be the smaller and greater is unlimited; and the unlimited is unknown; and the unknown itself cannot be a reason for other. | וידוע שהזויות הנצבות הם שוות לעולם מה שאין כן החדה והנרוחת כי יקבלו הפחות והיתר והדבר אשר יקבל הפחות והיתר הוא בלתי מוגבל והבלתי מוגבל הוא בלתי ידוע והבלתי ידוע בעצמו איך יהיה סבה לזולתו | |
| Finding the Area | ||
|---|---|---|
| The discussion on the measuring of the equilateral right-angled quadrangle [= square] | הדבור על מדידת המרובע שוה הצלעות נצב הזויות | |
| You should know in general that for every right-angled quadrangle, its area is the product of its one side by its other side. | ראוי שתדע בכלל כי כל בעל ארבעה צלעות נצב הזויות הארבעה הנה הכאת צלעו האחד בצלעו האחר ככה תשברתו | |
| This is the reason that the square of its one side is equal to the product of its one side by its other side [sic.]. | והיה זה סבה להיות שמרובע צלעו האחד שוה להכאת צלעו הא' בצלעו השני | |
|
דמיון מרובע אבג"ד הוא שוה הצלעות כי נשים כל א' מצלעותיו עשר אמות | |
|
והנה מרובע צלעו האחד מאה אמה וככה הוא תשברתו | |
|
כי הם מאה אמות מרובעות נצבות הזויות כל אמה מהן מרובעת דומה אל המרובע הגדול | |
| Proof: we divide each of its side by ten cubits with ten points and we draw a line from each point on one side to the side parallel to it. | המופת על זה נחלק כל צלע ממנו על עשר אמות בעשרה נקדות ונגיע מכל נקדה מהצלע האחד קו על הצלע הנכחי לו | |
|
הנה יהיו הקוים נכוחיים האחד לחברו ולצלעו המרובע הגדול כי מרחקי קצותיהם אשר הם על זויות נצבות שוים | |
|
אם כן קו ה"ו נכחי אל קו א"ד וקו כ"ל נכחי אל קו א"ב | |
|
ונאמר תחלה כי קו א"ד נפל על שני קוי א"ב וכ"ל הנכוחיים | |
|
הנה ישרת שתי הזויות הפנימיות אשר בצד אחד שוות לשתי נצבות | |
|
|
וזוית א' היא נצבת | |
|
אם כן גם זוית כ' היא נצבת על כל פנים | |
|
[128]והתמונות הנכחיות הצלעות יהיו זויותיהם המומרות שוות | |
|
א"כ גם זוית ה' נצבת | |
|
וגם הזוית הנשארת מזה המרובע הקטן שהוא האמה נצבת | |
|
עוד קו ו"ה נפל על קו א"ב | |
|
והקו הישר אשר יפול על קו ישר הנה ב' הזויות אשר בצדו אם נצבות או שוות לשתי נצבות | |
|
וזוית ה' נצבת אם כן גם הזוית אשר בצדו נצבת | |
|
ומזה יתבאר כי גם על ד' זויות האמות נצבות וכן יתבארו כל הי' אמות אשר בטור הא' | |
|
עוד קו כ"ל נחתך בקו ה"ו | |
|
וב' הקוים אשר יחתכו זויותיהם המקבילות שוות | |
|
א"כ גם זוית האמה הראשונה אשר בטור הב' השמאלית נצבת וכנגדה נצבת | |
|
וכן יתבארו כל האמות אשר בתשבורת הזאת | |
|
ולהיותם שוי הצלעות יתבאר מחלוקת כל צלע השוה לחלקים וכשתחסר מן השוים יהיה הנשאר שוים והב' דברים השוים לדברים שוים גם הם שוים | |
| Rectangle | ||
| This measuring is true for the right-angled quadrangle [= rectangle], i.e. that you multiply its one side by its other side that are encompassing one angle [= adjacent sides] and find the area. | וזאת המדידה נכונה בבעלות ד' הנצבות הזויות הד' ר"ל שתכפול צלעו הא' על צלעו השני המקיפו' בזוית א' ולמצוא התשבורת | |
|
דמיון בבעלת ד' צלעות נצב הזויות הד' נכוחי הצלעות אשר צלעה הא' י' וצלעה האחרת ה' | |
|
כזה | |
|
הנה כפילת הה' על הי' הם נ' וזהו התשבורת של זאת התמונה | |
|
ומופתה כמופת הקודם | |
| Rhombus | ||
| However, if the shape is equilateral but not right-angled, it cannot be measured by its sides, because it leads to a huge error. | אבל אם היתה התמונה שוה הצלעות ולא היתה נצבת הזויות ולא יתכן שימדד במדידת צלעותיה כי זה יביא לטעות גדולה | |
|
דמיון יש לנו מרובע שוה הצלעות ובלתי נצב הזויות עליו אבג"ד ויהיה כל א' מצלעיו י' אמות | |
|
הנה אם תכפול צלעו הא' בצלעו השני אז אם תקח מרובע צלעו הא' יעלה ק' ואין זה תשבורת המרובע הזה | |
| Proof: we draw two diagonals from its vertices: one is diagonal AG and the other is diagonal BD. | המופת נוציא ב' קוטרים מזויותיו הא' קוטר א"ג והב' קטר ב"ד | |
|
ויהיה קטר א"ג י"ו אמה וקטר ב"ד י"ב אמה | |
|
ויחתכו על נקדת ה' | |
|
הנה נעשה שטח נכוחי הצלעות נצב הזויות אשר צלעו אחת כקטר הארוך וצלעו האחרת כקטר הקצר | |
|
ויהיה כל צלע נכוחי לקטר הדומה לו כי שתי הקטרים יחתכו על זויות נצבות כאשר נראה המופת במדידתו | |
|
ונכתוב עליו ה' ו' ז' ח' | |
|
הנה נחלק השטח הנצב הזויות לד' חלקים שוים עם קטרי המרובע הקטן אשר אין זויותיו נצבות | |
|
עוד כל חלק מהד' חלקים נחלק לשני חלקים שוים עם צלעות המרובע הקטן לא פחות ולא יתר | |
|
ותשבורת השטח הנצב הזויות הוא בכפל צלעו האחד על השני | |
|
וצלעו הא' הוא י"ו אמה כי [129]הוא שוה לקטר הארוך מהמרובע | |
|
וצלעו השנית הוא י"ב אמה כי הוא שוה לקטר הקצר מהמרובע | |
|
וכפל י"ו על י"ב הם קצ"ב וזהו תשבורת השטח הנצב הזויות אשר הוא כפל תשבורת המרובע אשר בתוכו | |
|
ואם כן תשבורת המרובע הם צ"ו אמות אשר בתוכו | |
|
ואלו היינו נשענים על כפל צלעותיו היה יוצא ק' אמה וזאת שגיאה גדולה | |
| Therefore, the geometricians said that this quadrangle is measured by multiplying its one diagonal by the other diagonal and this is its area. | ולכן אמרו בעלי השעור שזה המרובע יהיה נמדד בכפילת מחצית קטרו הא' על קטרו השני והוא תשברתו | |
| The proof of this measurement: it is known that since it is equilateral, its two diagonals intersect at right angles, because each diagonal divides it into two [equal] isosceles triangles and the diagonal is the base of the two triangles. | המופת על המדידה הזאת ידוע שכאשר יהיה שוה הצלעות הנה שני קטריו יחתכו על זויות נצבות כי כל קטר הוא מחלק אותו לשני משולשים שוה השוקים והקטר תושבת שני המשולשים | |
| When one diagonal is drawn from one vertex of the isosceles triangle opposite to the base to the other vertex of the [other] isosceles triangle opposite to the base, it divides the base also into two equal parts at right angles. | וכאשר יצא הקטר האחד מזוית משולש שוה השוקים אשר התושבת היא מיתרה עד הזוית האחרת ממשולש שוה השוקים שזאת התושבת היא מיתר גם אותה תחלק התושבת לשני חלקי' שוים [130] ועל זויות נצבות | |
| The product of the whole diagonal by the whole diagonal is the area of the outer quadrangle that is its double. | וכפילת כל הקוטר בכל הקטר יהיה תשבורת המרובע החיצון אשר הוא כפלו | |
| So, the [product] of its half by its whole is half the area of this [outer] quadrangle. | לכן מרובע חציו בכולו הוא מחצית זה שהוא תשבורת המרובע הזה | |
| Therefore, they said that when you find an equilateral quadrangle, whether it is right-angled or not, you should measure it only by measuring the diagonals, so that you will never make a mistake. | ולכן אמרו כשתמצא מרובע שוה הצלעות בין שיהיה נצב הזויות בין שלא יהיה לא תמנה אותו כי עם מדידת האלכסונים ולא תטעה לעולם | |
| Because it is not easy for the person to identify whether the angle is right or not, and therefore one should be careful of making a mistake. | כי להכיר האדם הזוית הנצבת מהבלתי נצבת אינו בקלות ולכן ראוי להשמר מן הטעות | |
| Finding the Side | ||
| Square | ||
| If the quadrangle is a square and you do not know its side, or you wish to measure it by its one diagonal: | ואם היה המרובע שוה הצלעות נצב הזויות ולא ידעת צלעו או רצית למדדו מקטרו האחד | |
| Take its square and divide it into two [equal] parts: the one part is the area of that square and the square of the part is the length of its side. | תקח מרובעו ותחלקהו על שני חלקים והחלק האחד הוא תשבורת המרובע ההוא ושרש החלק הוא אורך צלעו | |
|
דמיון מרובע אשר עליו אבג"ד שוה הצלעות נצב הזויות והיה מרובע קטרו ר' | |
|
תחלק הר' לשני חלקים יהיה החלק הא' ק' והוא תשבורת המרובע | |
|
ושרש הק' שהם י' הם אורך צלעו | |
| Rectangle | ||
| If it is not equilateral, but it is right-angled, when its diagonal and one of the sides are known to you and you wish to measure it by these two: | וכן אם אינו שוה הצלעות אבל הוא נצב הזויות יהיה הקטר ידוע אליך והצלע האחד ורצית למדדו משתי אלה | |
| Take the square of the diagonal and subtract it from the square of the known side; the remainder is the square of the remaining side. | תקח מרובע הקטר ותגרע ממנו מרובע הצלע הידוע והנשאר הוא מרובע הצלע הנשאר | |
| Extract its root and it is the length of the side. | תקח שרשו והוא אורך הצלע | |
| Multiply it by the known side and it is the area of the square. | תכה אותו עם הצלע הידוע והוא תשבורת המרובע | |
|
דמיון מרובע ארוך אשר עליו אבג"ד והיה צלע א"ב י' אמות וקטר א"ג י"ב אמות | |
|
לקחנו מרובע הקטר והוא קמ"ד אמות גרענו ממנו ק' אמה שהוא מרובע הצלע הידוע שהוא י' אמות ונשארו מ"ד אמות ושרשם ו' אמות ושני שלישי אמה פחות משהו שהוא אורך הצלע השני | |
|
הכינו הו' ושני שלישי' פחות משהו עם הי' ונהיו ס"ו אמות ושני שלישים פחות משהו והוא תשבורת המרובע | |
| The proof of these two measurements: it is known that the diagonal divides these two quadrangles into two equal right triangles, since their sides are parallel and the diagonal is shared by both, in each triangle there is one right angle and the diagonal is the hypotenuse opposite to the right angle in each triangle. | המופת על שתי [131]על שתי אלו המדידות ידוע שהקטר הוא חילק את שני המרובעים האלה על שני משלשים שוים נצבי הזויות להיות שהם נכוחיי הצלעות והקטר משותף בשניהם וזוית אחת בכל משלש נצבת והקטר הוא מיתר הזוית הנצבת לכל משולש מהם | |
| It has already been clarified in geometry that [the sum of] the two squares of the sides surrounding the right angle in the right-angled triangle is equal to the square of the side that is the hypotenuse opposite to the right angle. | וכבר התבאר בחכמת המדות שהמשולש הנצב הזוית שני המרובעים אשר יהיו מהצלעות המקיפות בזוית הנצבת שוות למרובע ההוה מהצלע שהוא מיתר לזוית הנצבת | |
| Therefore, when you subtract one of them from the [sum of] the two the other remains and its root is the required. | א"כ כשתגרע מהשוה לשנים האחד ישאר השני ושרשו הוא המבוקש | |
| The result of multiplying it by the other that surrounds the right angle is the area of that quadrangle. | הנולד מכפילתו בחברו אשר יקיף עמו בזוית הנצבת הוא תשבורת המרובע ההוא | |
| If the area of the quadrangle is known to you and you also know one of its side, but you do not know its other side: | ואם היתה תשבורת המרובע ידועה לך וידעת גם צלעו האחד ולא ידעת גם צלעו השני | |
| Divide the area of the [rectangle] by the known side and you receive the other unknown side. | תחלק תשבורת המרובע על הצלע הידוע ויצא לך הצלע האחר הבלתי ידוע | |
|
דמיון היה מרובע תשברתו ק' אמה וצלעו האחד י' אמה | |
|
תחלק תשברתו שהוא הק' אמה על י' ויצא לך החלק י' ומזה ידעת שגם צלעו האחר י' אמות והמרובע הזה שוה הצלעות | |
|
וכן אם היה תשברתו ק' אמה וצלעו הא' כ' אמה | |
|
תחלק הק' על הכ' ויצא לך החלק ה' והוא צלעו האחר ומזה ידעת שהוא שטח נכחי הצלעות אשר יקרא מרובע ארוך | |
| Proof: the area is a number that is generated from the product of the parts of one side by the parts of the other side. | המופת על זה כי התשבורת הוא מספר נולד מכפילת חלקי הצלע האחד בחלקי הצלע האחר | |
| The number multiplied by another number is found in this product of the one number as the quantity of the other number and also the other [number] as this one. | והמספר הנכפל במספר אחר ימצא באותה תולדה המספר האחד בכמות המספר האחר וכן האחר בזה | |
| So, when you know the quantity of one number and the product, if you divide the product by the quantity of the known number, we know the number that is found [in the product] as the quantity of the known number. | וכשידעת כמות מספר האחד והתולדת אם תחלק התולדת על כמות המספר הנודע ידענו המספר אשר ימצא בכמות המספר הנודע | |
| The product is generated from its duplication as the times of the known number. | ובהתקבצו כפעמי המספר הנודע נהיה התולדת | |
| Therefore, we divide the area of the quadrangle by its known side and this allows us to know its unknown side. | ולכן חלקנו תשבורת המרובע על צלעו הנודע והוא יודיע לנו צלעו הבלתי נודע | |
| Rhombus | ||
|
ואם היה המרובע שוה הצלעות ובלתי נצב הזויות והוא אשר יקרא מעויין והיה קטרו הא' י"ו אמה וקטרו הב' י"ב אמה כמה יהיה צלעו | |
| Divide each diagonal into two [equal] parts and take the square of one part of each. | תחלק כל קטר לשני חלקים וקח מכל אחד מרובע החלק האחד | |
| Sum up the two squares as one number, extract the root and it is the side of that quadrangle. | ותחבר שני המרובעי' כאלו הם מספר אחד וקח השרש והוא צלע המרובע ההוא | |
|
דמיון חלקנו קטר י"ו על ח' ח' ולקחנו מרובע ח' שהם ס"ד וכן קטר הי"ב על ו' ו' ולקחנו מרובע הו' שהם ל"ו חברנו הס"ד עם הל"ו ונהיו ק' ושרשו י' והוא צלע המרובע | |
|
המופת על זה כי כל צלע מצלעי זה המרובע יהיה קטר לשטח נכחי הצלעות נצב הזויות אשר צלעו הא' ח' וצלעו השני ו' כאשר הודענו במדידת המרובע הזה | |
|
ומרובע הקטר ראוי להיותו שוה לשני מרובעי כי הוא מיתר למשלש בזויתו הנצבת ולכן ישוה זה צלע המרובע לשרש [132] שני מרובעי חציי קטריו | |
|
ואם שאל השואל מעויין שקטרו האחד [133]שקטרו האחד י"ו אמה וצלעו י' אמה כמה קטרו האחר | |
|
תקח מרובע מחצית הקטר הידוע שהוא ח' ומרובעו ס"ד ותגרעהו ממרובע הצלע שהוא ק' ונשאר שהוא ל"ו הוא מרובע חצי הקטר האחר תקח שרשו והוא ו' והוא מחצית הקטר כפלהו והוא י"ב והוא הקטר האחר | |
|
| ||
| Proof: it is known that half of each of the two diagonals stands on the other diagonal at right angle. | המופת על זה ידוע שמחצית כל קטר משניהם עומד על הקטר האחר על זויות נצבות | |
| Half of the known diagonal with half of the unknown diagonal and the side of the quadrangle form a right-angled triangle, such that the side is the hypotenuse opposite to right angle. | ומחצית הקטר הידוע עם מחצית הקטר הבלתי ידוע וצלע המרובע יעשו משלש נצב הזויות והצלע יהיה מיתר הזוית הנצבת | |
| The square of the side, which is the hypotenuse opposite to right angle, is equal to [the sum of] the squares of the two remaining sides, which are half the diagonals. | ומרובע הצלע שהוא מיתר לזוית הנצבת ישוה לשני מרובעי שתי הצלעות הנשארות שהם חציי הקטרים | |
| When you subtract the square of the one side surrounding the right angle, which is half the diagonal here, from the square of the hypotenuse opposite to right angle, the square of half the other diagonal that surrounds the right angle remains and its root is half the diagonal. | וכאשר תגרע מרובע הצלע הא' המקיף בזוית נצבת אשר הוא בזה מחצית הקטר ממרובע צלע המרובע שהוא המיתר לזוית הנצבת ישאר מרובע חצי הקטר האחר שהוא המקיף האחר לזוית הנצבת ושרשו הוא חצי הקטר | |
| If you double it, you find the whole diagonal. | ואם תכפלהו תמצא כל הקטר | |
| As such questions you can also draw other questions and answer them. | ובכאלה השאלות תוכל להוציא גם אתה שאלות אחרות ולהשיב עליהן | |
| Here comes the explanation of the measuring of various triangles, then we explain the measuring of the trapezoids, because we cannot explain the measuring of the trapezoids before the measuring of the triangles, since the triangles are basis of the trapezoids. | ומהנה נכנס בבאור מדידת המשולשי' למיניהם אחר נבאר מדידת הנוטים כי לא נוכל לבאר מדידת הנוטים ראשונה למדידת המשולשים אחר שהמשולשים הם יסוד לנוטים | |
| For, every trapezoid is divided into two different triangles or more. | כי כל נוטה יחלק לשני משולשים מתחלפים או ליותר מזה | |
| So, when you know the measuring of the different triangles, you can easily gain the knowledge of measuring the trapezoids. | וכאשר תדע מדידת המשולשים למיניהם יגיע לך בקלות ידיעת מדידת הנוטים | |
Chapter Three on the Measuring of the Various Triangles |
הפרק השלישי במדידת המשולשים למיניהם | |
| You already know that the triangles are divided into equilateral, isosceles, and scalan triangles. | כבר ידעת שהמשולשים נחלקו לשוי הצלעות ולשוי השוקים ולמתחלפי הצלעות | |
| We will first explain the methods of the equilateral triangle, because the height falls in it on half the base, for any side you wish to extract; then we will discuss the other triangles. | ונקדים לבאר תחלה דרכי המשלש השוה הצלעות כי בו יפול העמוד במחצית התושבת בכל צלע שתרצה להוציאו אחר נדבר על המשלשים האחרים | |
| We first say that the common thing for the measuring of all the triangles is that you multiply the height by half the base, or the whole base by half the height and the result is the area of the required triangle. | ונאמר בתחלה כי הדבר הכולל לכל המשלשים במדידתם הוא שתכפול העמוד במחצית התושבת או כל התושבת במחצית העמוד וההוה הוא תשבורת המשלש המבוקש | |
| Therefore one should first state how the height is found in each triangle, so that it becomes known, because with it we receive the unknown area of the triangle. | ומפני זה ראוי להודיע בתחלה איך יצא העמוד בכל משלש ומשלש עד שיהיה ידוע כי בו יגיע לנו תשבורת המשלש המוסכלת | |
| Equilateral triangle and isosceles triangle | ||
| We say that in the equilateral triangle the height always falls on half the base, since its three angles are always acute angles and its three sides are equal. | ונאמר כי במשלש השוה הצלעות לעולם יפול העמוד במחצית התושבת אחר שג' זויותיה הם חדות לעולם וג' צלעותיה שוות | |
| By height I mean the line that falls from the vertex at the top of the triangle to its base at right angle, which is called the height of the shape. | וארצה בעמוד הקו הנופל מזוית ראש המשלש אשר יקרא גובה התמונה על תושבתה על זויות נצבות | |
|
המשל בזה משלש אב"ג שוה הצלעות ועמוד אשר יצא מראש המשלש אשר הוא נקדת א' על תושבתה יפול בנקדת ד' ואומר שנקדת ד' היא מחצית התושבת | |
| Proof: the height divides the triangle into two triangles, two sides of each of these triangles are equal to two sides of the other, each to its corresponding: | המופת בזה העמוד חולק את המשלש לשני משלשים אשר שתי צלעות כל משולש ממנו שוות לשתי הצלעות מהאחר כל אחד [134]לגילו | |
|
|
צלע א"ג שוה לצלע א"ב | |
|
ועמוד א"ד צלע משותף לשתיהן | |
|
וזוית אד"ב שוה לזוית אד"ג כי שתיהן נצבות | |
|
אם כן צלע ג"ד שוה לצלע ד"ב | |
|
וצלע ג"ד וד"ב הם מחוברים הם כל התושבת אם כן כל אחת מהן היא מחצית התושבת | |
| The proof that the area of every triangle is the product of the height by half the base or of the whole base by half the height, whether it is an equilateral triangle, or an isosceles triangle, whose height is drawn on its different side: | והמופת אשר תשבורת כל משלש הוא כפילת העמוד בחצי תושבת או כל התושבת בחצי העמוד אם במשולש שוה הצלעות או במשלש שוה השוקים אשר הוצאת עמודו על צלעו המתחלפת | |
| The same proof that applies to the equilateral triangle, applies to the isosceles triangle, whose height is drawn on its different side. | הוא זה כי המופת אשר הורה במשלש שוה הצלעות הוא בעצמו יורה זה על שוה השוקים כאשר הוצאת עמודו על צלעו המתחלפת | |
| Because, when you draw the height on half the base, then you draw a line at the end of the base parallel to the height and you draw also a line from the top of the height parallel to half the base, a rectangle is formed, whose one side is the height and its other side is half the base. | כי כאשר הוצאת העמוד על מחצית התושבת אח"כ הוצאת קו על קצה התושבת נכחי אל העמוד עוד הוצאת קו מראש העמוד הנכחי אל מחצית התושבת כבר נהיה שטח נכחי הצלעות אשר צלעו האחת העמוד וצלעו האחר מחצית התושבת | |
| [The area of] this surface is equal to [the area of] the mentioned triangle, for the side of the mentioned triangle is a diagonal of the mentioned rectangle that divides it into two equal triangles, such that one triangle is half the whole mentioned triangle. | והשטח הזה שוה למשלש הנזכר כי צלע המשלש הנז' הוא קטר לנכחי הצלעות הנז' ומחלק אותו לשני המשלשים שוים והמשולש האחד הוא מחצית כל המשלש הנזכר | |
| Therefore the whole rectangle divided into two [equal] triangles is equal to the whole mentioned triangle. | אם כן כל שטח הנכחי הצלעות אשר הוא נחלק לשני משולשים הוא שוה לכל המשלש הנז' | |
| The area of the rectangle is the product of its one side by its other side. | ותשבורת השטח הנכחי הצלעות הוא כפילת צלעו האחת בצלעו האחרת | |
| Its one side is the height of the triangle and its other side is half the base of the triangle. | וצלעו האחת היא עמוד המשלש וצלעו האחרת היא מחצית תושבת המשלש | |
| Hence, the area of the triangle is the product of the height by half its base, since it is equal to [the area of] the rectangle. | אם כן תשבורת המשלש הוא כפילת העמוד בחצי תושבתו אחר שהוא שוה לשטח הנכחי הצלעות | |
|
המשל בזה משלש אב"ג הוא שוה הצלעות או השוקים ועמודו הוא קו א"ד והוצאנו על קצה מחצית תושבת המשלש הזה קו ב"ה על נקדת ב' נכחי לקו א"ד ועל נקדת ה' הוצאנו קו א"ה נכחי לקו ד"ב הנה אומר כי שטח א"דה"ב הנכחיי הצלעו' שוה למשלש אב"ג | |
|
המופת בזה כי זוית אה"ב שוה לזוית אד"ב כי שתיהן נצבות | |
|
והקטר שהוא צלע המשלש שהוא א"ב חלק את השטח לשני משלשים שוים אחר שהוא קו ישר ונפל על שני קוים נכוחיים שם את שתי הזויות המומרות שוות | |
|
אם כן זוית ב"ד היא שוה לזוית בא"ה | |
|
וקטר א"ב משותף | |
|
אם כן משלש הא"ב שוה למשלש אב"ד | |
|
ומשלש אב"ד הוא חצי שטח הנכחי הצלעות שהוא שטח ה"אד"ב והוא חצי משלש אב"ג | |
|
אם כן שטח ה"אב"ד שוה למשולש אב"ג | |
|
ושטח נכחי הצלעות הנצב הזויות כפילת צלעו האחת בצלעו האחרת היא תשברתו | |
|
אם כן גם תשבורת משלש אב"ג הוא כפילת צלע נכחי הצלעות האחת שהוא עמוד המשלש בצלעו האחרת שהוא מחצית תושבת המשולש | |
|
וזמש"ל | |
| The proof that by multiplying half the height by the whole base we find the area is, if you want, from the known rule that for every number, the product of its half by a whole other number is as the product of its whole by half that number. | ואם המופת שבכפילת מחצית העמוד על [135]על כל התושבת נמצא התשבורת הוא זה אם תרצה מהכלל הידוע שכל חשבון כפולת חציו בחשבון אחר כלו הוא ככפולת כלו כמחצית אותו החשבון | |
| If you want, we provide proof in geometry: | ואם תרצה נביא מופת מחכמת המדות והוא זה | |
|
משלש אב"ג שוה הצלעות | |
|
ונוציא מנקדת א' ממנו עמוד אל תושבת ב"ג והוא קו א"ד | |
|
ונסמן במחציתו נקדת ה' | |
|
ונוציא מנקדת ה' שני קוים דבקים על יושר נכוחיים ושוים לתושבת ב"ג והם ה"ז ה"ח | |
|
ונגיע קוי ח"ב ז"ג | |
|
הנה אומר כי שטח ב"ג ז"ח הנכחיי הצלעות שוה למשלש אב"ג | |
|
המופת כי נוטה ט"כב"ג משותף לשטח ב"גז"ח ולמשלש אב"ג | |
|
ונשאר משלש חט"ב כמשלש אט"ה | |
|
כי זוית חט"ב שוה לזוית הט"א | |
|
|
וזוית אה"ט שוה לזוית בח"ט | |
|
וצלע א"ה שוה לצלע ב"ח כי שתיהם מחצית העמוד | |
|
אם כן משלש חט"ב שוה למשלש אט"ה | |
|
וכן משלש כז"ג שוה למשלש אכ"ה | |
|
וההנהגה אחת | |
|
א"כ נוטה ט"כב"ג עם שני משולשי חט"ב כז"ג שהם כל השטח נכחי הצלעות שוה לנוטה ט"כב"ג עם שני משולשי אט"ה אכ"ה שהם כל משלש אב"ג | |
|
אם כן משלש אב"ג שוה לשטח נכחי הצלעות ב"גז"ח | |
|
ותשבורת השטח הנכחי הצלעות הוא הכאת צלעו האחד שהוא שוה למחצית העמוד של המשולש בצלעו האחר שהוא שוה לכל תושבת המשולש | |
|
אם כן גם תשבורת המשולש שהוא שווה לו הוא הכאת מחצית העמוד בכל התושבת | |
|
וזה מש"ל | |
| This same proof applies to the isosceles triangle when you draw the height on its different side. | וכן זה המופת בעצמו יורה על משלש שוה השוקים כאשר הוצאת העמוד על צלעו המתחלפת | |
| You already know that when you have an equilateral triangle or an isosceles triangle, you multiply the height by half the base, or vice versa, then you find the area. | הנה כבר ידעת שכשיבא בידך הן משלש שוה הצלעות הן שוה השוקים תכפול העמוד במחצי' התושבת או ההפך ותמצא התשבורת | |
| In order to find the height from the side in an equilateral triangle or an isosceles triangle, do as follows: | ולדעת העמוד מהצלע הן במשלש שוה הצלעות או במשולש שוה השוקים תעשה כן | |
| Take the square of half the base, subtract it from the square of the side, then extract the root of what remains and this is the height. | תקח מרובע חצי התושבת ותגרעהו ממרובע הצלע ואשר ישאר יקח שרשו והוא העמוד | |
| Proof: the side of the triangle is the hypotenuse opposite to the right angle surrounded by the height and half the base. | המופת על זה כי צלע המשולש הוא מיתר הזוית הנצבת אשר יקיפו בה העמוד ומחצית התושבת | |
| It has already been clarified in geometry that the square of the hypotenuse opposite to the right angle is equal to [the sum of] the squares of the two sides surrounding it. Therefore, when you subtract the square of one side from the square of the hypotenuse opposite to the right angle, then extract the root of the remainder, you find the other side. | והתבאר בחכמת השעור כי מרובע הצלע אשר היא מיתר הזוית הנצבת [שוה לשני מרובעי צלעות המקיפות בה לכן כשתגרע מרבע הצלע האחת ממרובע המיתר הזוית הנצבת][136] ותקח שרש הנשאר תמצא את צלע האחרת | |
| However, the geometricians gave other rules of measuring the equilateral triangle, in order to make it easier for the one who calculates, and said: always take from 15 [parts of] the side its 13 [parts] and this is the height; or square the side, subtract its quarter from it, then extract the root of the three-quarters and this is the height of this triangle. | אולם בעלי השעור נתנו כללים אחרים במדידת המשולש השוה הצלעות כדי להקל על המחשב ואמרו לעולם תקח מט"ו בצלעו י"ג והוא העמוד או תהיה מרבע את הצלע והוצא ממנו הרביעית וקח [שרש השלש רביעיות][137]והוא עמוד המשולש הזה | |
| These rules and their like are derived from the aforementioned proof. | ואלה הכללים וכדומה להם יצאו מהמופת הנז' | |
| What we have said regarding the isosceles triangle is when you draw its height one its different side, but if you draw it on one of its legs, know that it has two other rules that we will explain when we will discuss the scalene triangle. | ומה שאמרנו בענין משלש שוה השוקים הוא כשהוצאת עמודו על צלעו המתחלפת אבל אם הוצאת אותו על צלע אחד מצלעותיו דע שיש לו שני משפטים אחרים נבארם כשנבאר [138]משפטי המשלש שמתחלף הצלעות | |
| Scalene triangle | ||
| If we wish to measure a scalene triangle, we should extract its height on its side first and to know its distance from one of the sides, for the height that we extract in the equilateral triangle and in the isosceles triangle always falls on the middle of the base, yet this height that is drawn in the scalene triangle does not fall on the middle [of the base] at all, but it is far from one side and close to the other side. | אם נרצה לשער המשלש המתחלף הצלעות תחלה ראוי להוציא עמודו על צלעו המתחלפת ולדעת מרחקו מאחת הצלעות כי העמוד אשר הוצאנו במשלש השוה הצלעות ובמשולש שוה השוקים הוא נופל במחצית התושבת לעולם אבל אין העמוד הזה היוצא במשולש המתחלף הצלעות יוצא על המחצית כלל אבל הוא רחוק מהצלע האחת והוא קרוב אל הצלע השנית | |
| We call its distance from the first side "the close distance" and we call its distance from the far side "the far distance". | ומרחקו מהצלע האחת אנו קוראים אותו המרחק הקרוב ומרחקו מהצלע הרחוק אנו קוראים אותו המרחק הרחוק | |
| After we know the position of the height on one of the sides, we can then announce the length of its height. | ואחר שנדע גבול מעמדו באחת מהצלעות אז נביא להודיע אורך עמודו | |
|
ונאמר משלש אב"ג מתחלף הצלעות אשר צלע א"ב ממנו עשרים אמה וצלע א"ג ממנו י"ח אמה וצלע ג"ב ממנו אשר עליו נוציא את העמוד והוא התושבת י"ו אמה | |
|
ונרצה לדעת גבול מעומד העמוד מצלע א"ג אשר הוא הקצר | |
|
וכבר ידענו שאורך הצלע הזה י"ו אמה | |
|
נקח מרובעו הוא שכ"ד | |
|
ונחבר אליו מרובע התושבת שהוא רנ"ו ויהיו תק"ף | |
|
אחר נגרע מן תק"ף מרובע א"ב אשר הוא הצלע הארוך וכבר ידענו שהוא כ' ומרובעו הוא ת' נגרעם מן תק"ף נשארו ק"פ | |
|
נקח מחציתם והם צ' | |
|
ונחלק אותם על התושבת שארכה י"ו ונהיה החלק הא' ה' וחצי ושמינית וזהו גבול מעמד העמוד מן הצלע הקצר על התושבת | |
|
| ||
|
ואם רצינו לדעת גבול מעמדו מן הצלע הארוך | |
|
נקח מרובעו שהוא ת' ונחבר אותו עם מרובע התושבת שהוא רנ"ו ושניהם תרנ"ו | |
|
נגרע מאלה מרובע הצלע הקצר שהוא שכ"ד נשארו של"ב | |
|
נקח מחציתם והם קס"ו | |
|
ונחלקם על התושבת שהם י"ו ונהיה י' ושליש וחלק אחד מכ"ד וזהו גבול מעמד העמוד מן הצלע הארוך על התושבת | |
|
| ||
|
עוד נמשיל במשלש מתחלף הצלעות משל אחד על חשבון שלם ר"ל שיצא גבול מעמד עמודו על התושבת באחדים שלמים מבלתי חלק | |
|
ונאמר משלש אב"ג אשר צלע א"ג ממנו י"ג וצלע א"ב ממנו ט"ו וצלע ב"ג ממנו אשר הוא התושבת י"ד | |
|
ורצינו לדעת גבול מעמד העמוד על התושבת מהצלע הקצר שהוא א"ג ממנו | |
|
חברנו אל מרובע הצלע הזה מרובע התושבת ונהיו שס"ה | |
|
גרענו מזה המרובע מהצלע הארוך אשר הוא רכ"ה ונשארו ק"מ | |
|
לקחנו חצים והם ע' | |
|
חלקנום על י"ד שהוא חותך התושבת והיה החלק האחד ה' וזהו גבול מעמד העמוד מן הצלע הקצר | |
|
| ||
|
ואם תרצה לדעת גבול מעמדו מן הצלע הארוך | |
|
נחבר מרובעו עם מרובע התושבת ונהיו תכ"א | |
|
הוצאנו מהם מרובע הצלע הקצר שהוא קס"ט נשארו רנ"ב | |
|
לקחנו חצים והם קכ"ו | |
|
וחלקנום על י"ד שהוא התושבת היה החלק הא' ט' וזהו גבול [139]מעמד העמוד מן הצלע הארוך | |
|
| ||
| The proof of this has already been clarified in geometry, that the square of the side, which is opposite an acute angle, is less than the sum of the squares of the two other sides by double the rectangle that is encompassed by the whole base and the distance from the position of the height to that side. | המופת על זה כבר התבאר בחכמת השעור שמרובע הצלע אשר הוא מיתר זוית חדה הוא פוחת מן מרובעי שתי צלעות הנשארות בכפל שטח הנצב הזויות אשר יקיף בו התושבת כלו עם מקום מעמד העמוד מאותו הצד | |
| Therefore, when you add the square of the side to the square of the base and subtract the square of the other side from this, the remainder is double the rectangle that is encompassed by the whole base and the distance from the position of the height to that side. | ולכן כאשר תחבר מרובע הצלע עם מרובע התושבת ותוציא מהם מרובע הצלע הנשאר ישאר ככפל הנצב הזויות אשר יקיף בו כל התושבת והחלק אשר יצא עליו העמוד מאותו הצד | |
| Thus, we divide it by two and then divide by the base, as the sum is a double, and the result is the distance from the position of the height to that side. | ולכן נחלק אותו לשנים והחלק האחד נחלקהו על התושבת אחרי שהסך הוא כפל ואשר יצא הוא מקום מעמד העמוד על אותו הצד | |
|
המשל בזה המשלש אשר ציירנו | |
|
ידענו שצלע א"ג ממנו הוא י"ג אמה ומרובעו קס"ט | |
|
והתושבת י"ד אמה ומרובעה קצ"ו | |
|
ושתי המרובעים שס"ה | |
|
וצלע א"ב אשר הוא ט"ו אמה ומרובעו רכ"ה | |
|
יהיה פוחת משני המרובעים הנזכרי' בכפל השטח הנצב הזויות אשר יקיף בו ג"ד בג"ב | |
|
| ||
|
א"כ כאשר גרענו רכ"ה משס"ה ישאר ק"מ והוא ככפל השטח הנצב הזויות אשר יקיף בו ג"ד בג"ב | |
|
ומפני שהוא כפל לקחנו חציו והוא ע' והוא כשטח הנצב הזויו' אשר יקיף ג"ד בג"ב | |
|
חלקנוהו על התושבת שהוא י"ד וידענו שהחלק האחד ממנו הוא מקום מעמד העמוד מאותו הצד והחלק הוא ה' | |
|
ומזה ידענו שמרחק העמוד מאותו הצלע הוא ה' | |
| After we have determined the position of the height, we can calculate its length. We do as follows: | ואחר שידענו גבול מעמדו נבא לדעת ארכו וכן נעשה | |
| We subtract the square of the distance of the height on the base from the square of the adjacent side, so the remainder is the square of the height. | נגרע מרובע החלק מן התושבת אשר עומד עליו העמוד ממרובע הצלע הדבק בו ואשר ישאר הוא מרובע העמוד | |
|
המשל בזה המשלש | |
|
גרענו מרובע ה' שהוא כ"ה ממרובע הצלע הדבק בו[140] שהוא קס"ט [ונשארו קמ"ד][141] ושרשו י"ב והוא אורך העמוד | |
|
וכן אם רצינו לדעת אותו מהחלק האחר מהתושבת אשר הוא ט' ומרובעו פ"א גרענו אותו ממרובע הצלע הדבק בו אשר הוא רכ"ה נשארו קמ"ד ושרשו י"ב והוא אורך העמוד | |
| Proof: the square of each side of them is equal to the square of the height and [the square of] the part of the base that is next to it, because each of them is the hypotenuse opposite to the right angle in its triangle. | והמופת על זה כי מרובע כל צלע מהם שוה עם מרובע העמוד והחלק מן התושבת הדבק בו מפני שהוא מיתר לזוית הנצבת כל א' במשלשו | |
| For, when you draw the height, the triangle is divided into two triangles. | כי כאשר הוצאת העמוד כבר נחלק המשלש לשני משלשים | |
| When you subtract the square of the part of the base from the square of the side next to it, the square of the height remains from the square of the side; and when you extract its root, you find the length of the height. | וכאשר גרעת מרובע החלק מן התושבת וממרובע הצלע הדבק בו נשאר במרובע הצלע מרובע העמוד וכשלקחת שרשו מצאת אורך העמוד | |
| We have explained the measurement of all types of triangles and their differences in terms of the sides. | הנה בארנו מדידת כל המשלשים למיניהם והבדלם מצד הצלעות | |
| Although the scalene triangles are included in these measurements. For, the isosceles triangle is necessarily either acute angled, right-angled, or obtuse angled, and likewise the scalene triangle. Yet, since they have special measures from the aspect of the angles also, we shall explain them, eventhough it is not necessary. | ואעפ"י שבמדות האלה יכללו גם מתחלפי הזויות כי המשלש שוה השוקים לא ימלט מהיותו חד הזויות ואם נצב הזויות או נרוח גם ככה המתחלף הצלעות אמנם בעבור שיש להם מדות מיוחדות [142]גם מפני הזויות נבארם ואין זה צורך | |
| Right-angled triangle | ||
| We say concerning the right-angled triangle that there is no need to draw its height to its hypotenuse opposite to the right angle, because each of its two sides surrounding the right angle are its heights. | ונאמר כי המשלש הנצב הזויות אין צורך להוציא עמודו על צלעו שהוא מיתר הזוית הנצבת כי כל אחת משתי צלעותיו המקיפות בזוית הנצבת הן עמודיו | |
| Therefore, if you multiply one of the mentioned sides by its other side surrounding the right angle, you find its area. | ולכן אם תכה אחד מהצלעות הנזכרות בצלעו האחרת המקפת עמו הזוית הנצבת תמצא תשברתו | |
| The proof of this is known from what we explained concerning the previous triangles. | והמופת על זה ידוע ממה שבארנו במשלשים הקודמים | |
| Obtuse triangle | ||
| Also with regard to the obtuse triangle, it is not necessary to draw its height to its side opposite to the obtuse angle, but if you wish to draw it, I will show you this way: | עוד המשלש הנרוח הזוית אין הכרח להוציא עמודו על צלעו שהוא מיתר הזוית הנרוחת לבד אבל אם רצית להוציאו בדרך זו אשר אורה לך | |
| It has already been clarified in geometry that the square of side opposite to the obtuse angle exceeds [the sum of] the squares of the two sides surrounding it by twice the product of the base of this side by the line that is drawn outside [the triangle] from the end of the mentioned base to the place where the height falls. | כבר התבאר בחכמת השעור שמרובע מיתר הזוית הנרוחת עודף על מרבעי שתי הצלעות המקיפות בה בכפל התושבת אשר עליה הצלע בקו היוצא חוצה מקצה התושבת הנזכרת עד מקום נפילת העמוד פעמים | |
|
המשל בזה משלש אב"ג נרוחת הזויות וזוית אג"ב ממנו היא הנרוחת אומר שמרובע א"ב שהוא מיתר הזוית הנרוחת עודף על מרובע צלע א"ג וג"ב בכפל ג"ב בג"ד פעמים | |
|
ודרך הוצאת העמוד הזה במשלש הזה כך | |
|
נשים הזוית הנרוחת אשר עליה ג' | |
|
וצלע ג"א ד' אמות וצלע ב"ג י"ג אמה וצלע א"ב שהוא מיתר הזוית ט"ו אמה | |
|
ומרובע ט"ו מוסיף על מרובע י"ג ומרובע ד' אשר הם קפ"ה אמה מ' אמה | |
| If you divide half the excess by one of the sides, you receive the distance of the height from that side. | ואם תחלק מחצית התוספת על אחת מהצלעות יצא לך מרחק העמוד חוצה מן הצלע ההוא | |
|
עד"מ חלקנו מחצית המ' אשר הוא התוספת והם כ' על קו ג"א אשר הוא ד' אמות ויצא החלק ה' וידענו מזה שהעמוד נפל חוצה מן צלע ג"א ה' אמות והוא קו ג"ד והעמוד עליה עמוד א"ד | |
|
ואם תחלק אותו על קו ב"ג אשר ארכו י"ג אמה יצא החלק אחד ושבעה[143] חלקים מי"ג והוא מרחק העמוד היוצא חוצה מן ג"ב והעמוד עליו | |
Chapter Four on the Measuring of the Various Trapezoids |
הפרק הרביעי במדידת הנוטים למיניהם | |
| The trapezoid is a quadrangle shape that is not a square, not a rectangle, not a rhombus, and not a parallelogram. | הנוטה הוא תמונה בעלת ד' צלעות שאינה לא מרובע ולא נכחי הצלעות ארוך ולא מעויין ולא דומה למעויין | |
| They are of many types, although they are all included in four types: | והם מינים רבים אעפ"י שכלם נכללים בד' | |
|
יש מהם ג' ששתי צלעותיו המקבילות שוות והראש והתושבת בלתי שוות אבל הם נכחיות כזה | |
|
ויש מהם ששתי הצלעות שוות והראש והתושבת בלתי שוות ולא נכחיות כזה | |
|
ויש מהם ששתי הצלעות הדבקות מהם שוות האחת לחברתה כזה וכן השתי צלעות האחרות | |
|
ויש מהם חד הזוית כזה | |
|
ויש מהם שאין צלע הא' שוה עם האחר כזה | |
|
ויש מהם נצב [שתי][144] הזויות כזה | |
|
ויש מהם נצב הזוית האחת כזה | |
|
ויש מהם נרוח הזוית כזה | |
|
ויש מהם תמונות עקלקלות זולת אלו | |
| Therefore I should give you the way we need for measuring them. | לכן ראוי לך שאתן דרך הצריכה לנו במדידתם | |
| Isosceles trapezoid | ||
| I start with the trapezoid whose two sides are equal but not parallel, whose the top base and bottom base are not equal but are parallel. | ואחל מהנוטה אשר שתי צלעותיו שוות ואינם נכוחיות והראש והתושבת בלתי שוות אבל [145]הם נכוחיות | |
|
ונאמר אם היה נוטה אבג"ד וצלע א"ב ח' אמות וצלע ד"ג י"ח אמות ושני צלעי א"ד ב"ג כל אחד מי"ג אמה ותרצה למצוא תשברתו | |
| Do as follows: | תעשה כך | |
| Subtract the top base from the bottom base, take half the remainder, then count that much from one vertex of the bottom base and mark a dot there. | תגרע הראש מהתושבת ומהמותר תקח מחציתו ומנה כמה הוא מזוית התושבת האחת וסמן שם נקדה | |
| Count also that much from the other vertex of the bottom base and mark a dot there. | עוד מנה כמה הוא מזוית התושבת האחרת וסמן שם נקדה | |
|
דמיון גרענו הח' מן הי"ח ונשארו י' | |
|
לקחנו מחציתם והם ה' | |
|
מנינו כן מזוית ג' וסמננו שם נקדת ז' | |
|
עוד מנינו כן מזוית ד' וסמננו שם נקדת ה' | |
|
הגענו א"ה וב"ז וידענו עמודי זאת התמונה | |
|
רצינו לדעת ארך העמודים | |
|
וכפלנו הצלע שהם י"ג על עצמם והיו קס"ט | |
|
הוצאנו מהם כ"ה שהוא מרובע ה' נשארו קמ"ד | |
|
לקחנו גדרם והם י"ב | |
|
וידענו שארך העמוד הם י"ב | |
|
חברנו הראש עם התושבת והם כ"ו | |
|
ולקחנו מחציתם והם י"ג | |
|
וכפלנום עם העמוד שהם י"ב והיו קנ"ו וזהו תשבורת הנוטה הזה | |
|
המופת על זה | |
|
ידוע שמחצית העודף יהיה מהצד האחד ומחציתו מהצד האחר עד שיוציאו העמודים עליהם | |
|
וצלע א"ד הוא מיתר הזוית הנצבת | |
|
ולכן מרבעו שוה למרובע העמוד עם תושבת משלש אד"ה | |
|
ותושבת משלש אד"ה ה' | |
|
ומרבעו כ"ה | |
|
תגרעהו ממרובע הצלע אשר הוא קס"ט נשאר קמ"ד | |
|
וגדרו י"ב והוא ארך העמוד | |
|
וידוע שתשבורת משלש אד"ה הוא כפילת העמוד בחצי ד"ה | |
|
וכן תשבורת משלש בז"ג הוא כפילת העמוד בחצי ז"ג | |
|
אם כן תשבורת שני משולשים האלה הוא כפילת העמוד בכל תושבת ד"ה | |
|
ותשבורת השטח הנצב הזויות והוא שטח אבה"ז הנה הוא כפילת העמוד בתושבת ה"ז | |
|
אם כן כפילת ד"ז עם העמוד הוא תשבורת נוטה אבג"ד | |
|
ויתבאר גם כן זה בצורה אחרת מהמופת | |
|
והוא יש לנו נוטה אבג"ד שוה השוקים וצלע א"ב שוה לצלע ג"ד וכל אחת מהן בעלת י"ג אמות וצלע א"ג ו' אמות וצלע ב"ד י"ו אמות ונרצה למצוא התשבורת והעמוד | |
|
אוציא קו נכחי א"ה לקו ג"ד | |
|
ואוציא עמוד א"ז על קו ב"ד | |
|
אם כן שטח אהג"ד הוא נכחי הצלעות | |
|
וא"כ א"ב שוה לה"ד | |
|
|
וג"ד לא"ה | |
|
ולפי שב"ד י"ו אמו' וה"ד ו' אמו' א"כ ב"ה הנשארת תהיה י' אמות | |
|
ולפי שמשלש אב"ה שוה השוקים וכל אחת מצלעותיו ידועה יהיה א"כ גם עמוד א"ז ידוע והוא י"ב אמות כאשר קדם ידיעתו | |
|
ונחלק צלעי א"ב ג"ד באמצע על נקדת ח"ט | |
|
ונוציא עמוד כח"ל מט"נ על קו ב"ד | |
|
אם כן משלש אכ"ח שוה למשלש בח"ל | |
|
|
ומשלש דמ"ט שוה למשלש גנ"ט | |
|
לכן אם נוסיף בשתוף בעל שש צלעות א"ח ל"נ ט"ד ישוה שטח כ"ל מ"נ הנכחי הצלעות לנוטה אבג"ד | |
|
ולפי שא"כ שוה לב"ל | |
|
וד"מ שוה לג"נ | |
|
אם כן א"כ ד"מ שוים לב"ל נ"ג | |
|
וכשיתוסף עליהם בשתוף א"ד ל"נ יהיו שני' כ"מ ל"נ שהם שני פעמים כ"מ שוה לשני [146]א"ד ב"ג | |
|
ושני א"ד ב"ג ידועים כי הם כ"ב אמות | |
|
א"כ גם כ"מ פעמים כ"ב | |
|
ויהיה כ"מ י"א אמות | |
|
אבל גם כ"ל י"ב אמות | |
|
ונוטה אבג"ד שוה לשטח כ"ל מ"נ הנכחי בצלעות | |
|
א"כ יהיה תשברתו קל"ב אמות כתשבורת הנכחי הצלעות | |
| Acute trapezoid | ||
|
ואם רצית למדוד נוטה חד הזוית כגון נוטה אבג"ד והזוית אשר אצל ב' חדה וצלע א"ב י"ג אמות וצלע ג"ד כ' אמות וצלע א"ד ו' אמות וצלע ב"ג כ"ז אמות ותרצה למצוא התשבורת והעמוד | |
|
כך תעשה | |
|
תגרע הו' אמות מן הכ"ז אמות וישארו כ"א אמות | |
|
ובהיות צלעות משלש החד הזויות ידועות והם י"ג וכ"א וכ' יודע גם ארך א"ז העמוד שהוא י"ב אמות כמו שידענו זה מקודם | |
|
ותחבר עם הו' אמות כ"ז אמות ויהיה מחציתו י"ו אמות וחצי | |
|
כפלם עם העמוד ויהיו קצ"ח והם תשבורת הנוטה | |
|
והמופת על זה | |
|
אוציא קו א"ה נכחי לקו ג"ד | |
|
ואוציא א"ז עמוד | |
|
ולפי שא"ה כ' אמות וג"ה ו' אמות אם כן ב"ה הנשאר כ"א אמות | |
|
ולפי שקו א"ז העמוד במשלש אב"ה החד הזויות הוא י"ב אמות וצלעי א"ב ג"ד נחלקים באמצע על נקדת ח' וט' ובח"ל הוא העמוד גם מט"נ כמו שהראנו בצורה הזאת הקודמת לזה יראה שנוטה אבג"ד שוה לשטח כ"ל מ"נ נכחי הצלעות | |
|
|
וקוי ב"ג א"ד ביחד הם כפל כ"מ | |
|
וכ"מ הוא י"ו אמות וחצי | |
|
וכ"ל הוא י"ב אמות כי כן הוא קו א"ז | |
|
א"כ תשבורת הנוטה הזה הוא קצ"ח אמות | |
| Obtuse trapezoid | ||
|
אם רצית למדוד נוטה נרוח הזויות כמו נוטה אבג"ד והזוית אשר אצל ב' נרוחת והיה צלע א"ב י"ג אמות וצלע ג"ד כ' אמות וצלע א"ג ו' אמות וצלע ב"ד י"ז אמות | |
|
כזה | |
|
תגרע הו' אמות מהי"ז אמות וישארו י"א אמות | |
|
ובהיות צלעי המשלש נרוח הזוית ידועות י"ג וי"ז וכ' ימצא גם העמוד שהוא י"ב | |
|
תחבר הי"ז עם הו' הם כ"ג | |
|
ומחצית זה הם י"א וחצי | |
|
תכפלם על י"ב יהיו קל"ח והוא תשבורת הנוטה הזה | |
|
והמופת על זאת המדידה | |
|
אוציא עמוד א"ה | |
|
ואוציא קו א"ז נכחי לקו ג"ד | |
|
יהיה א"כ קו א"ז כ' אמות | |
|
וקו ז"ד ו' אמות | |
|
ואם כן קו ב"ז הנשאר יהיה י"א אמות | |
|
ולכן לפי שמשלש אב"ז הוא נרוח הזוית יהיה קו א"ה י"ב אמות | |
|
וכן לפי מה שהתבאר למעלה השטח אשר יהיה מב"ד א"ג ביחד ומא"ה הוא כפל נוטה אבג"ד | |
|
אם כן תשבורת הנוטה הזה הוא קל"ח אמות | |
| Scalene trapezoid | ||
|
ואם רצית למדוד נוטה אבג"ד ולא יהיה צלע מצלעותיה נכוחי והזוית אשר אצל ג' נצבת ויהיה כל צלע מצלעותיה ידוע אם צלע א"ב י"ג אמות ואם ב"ג י' אמות ואם ג"ד כ' אמות [147]ואם ד"א י"ז אמות כזה | |
|
תעשה כך | |
|
תכפול הי' אמות על הכ' אמות ויהיו ר' | |
|
וקח מחציתם והוא ק' | |
|
ועוד תכפול הי' על עצמם ויהיו ק' | |
|
והכ' על עצמם ויהיו ת' | |
|
וחברם ויהיו ת"ק | |
|
והי"ג על עצמם ויהיו קס"ט | |
|
חברם עם הת"ק ויהיו תרס"ט | |
|
גרע מהם מרובע י"ז וישארו ש"פ | |
|
קח חציים והוא ק"צ | |
|
כפלם על עצמם ויהיו ל"ו אלפי' וק' | |
|
חלקם על ת'[ק'] ויהיה החלק ע"ב וחמישית | |
|
תגרעם מן קס"ט ישארו צ"ו וחצי וחמישית ועשירית | |
|
| ||
|
כפלם על ת"ק ויהיו מ"ח אלף ות' | |
|
| ||
|
קח גדרם ויהיו רכ"א | |
|
וקח מחציתם ויהיה ק"י והוא תשבורת משלש אב"ד | |
|
| ||
|
אבל גם תשבורת משולש בג"ד ק' אמות | |
|
אם כן תשבורת נוטה אבג"ד ר"י אמות | |
|
המופת על המדידה הזאת | |
|
נגיע קו ב"ד | |
|
ואעמיד עליה עמוד קו א"ה | |
|
ולפי שכל אחד מצלעי ב"ג ג"ד הם ידועות וזוית שאצל ג' נצבת אם כן משלש בג"ד יהיה ידוע | |
|
ויהיה גם מרובע ב"ד ידוע והוא ת"ק אמות | |
|
אבל גם מרובע א"ב ידוע | |
|
א"כ מרובעי א"ב ב"ד ידועים והם יותר ממרובע א"ד | |
|
אם כן זוית אב"ד תהיה חדה | |
|
ואם כן מרובעי א"ב ב"ד גדולים ממרובע א"ד בכפל השטח הנצב הזויות אשר יקיפו בו קוי [ד"ב ב"ה | |
|
אם כן כפל השטח הנצב הזוית אשר יקיפו בו ד"ב ב"ד ידוע לכן גם השטח הנצב הזוית אשר יקיפו בו קוי ד"ב][148] א' ב"ה פעם אחת ידוע והוא גדר מרובע ב"ד על מרובע ב"ה | |
|
אם כן גם מרובע ד"ב על מרובע ב"ה ידוע | |
|
ומרובע ב"ד ידוע | |
|
א"כ גם מרובע ב"ה ידוע | |
|
אבל גם מרובע ה"א על מרובע ב"ד וגדרו הוא השטח אשר יקיפו ב"ד [ד"ה][149] | |
|
א"כ גם השטח אשר יקיפו בו ב"ד ד"ה ידוע והוא כפל משלש אב"ד | |
|
אם כן גם משלש אב"ד ידוע | |
|
אבל גם משלש בג"ד ידוע | |
|
ולכן כל בעלת ד' צלעות אבג"ד ידועה | |
|
והיות גם העמוד היוצא מנקדת א' על קו [ג"ד][150] ידוע הנה אני מביא עליו המופת | |
|
יהיה נוטה עליו אבג"ד וכל אחת מצלעותיו תהיה ידועה וזוית בג"ד נצבת | |
|
והיות העמוד היוצא מנקדת א' על קו ג"ד ידוע אוציא אם על קו ג"ד עמוד א"ז ועל קו א"ז עמוד ב"ח ועל קו ב"ד עמוד א"ה | |
|
והוא מבואר כי קו ב"ד ידוע וא"ה העמוד אשר עליה לפי שגם ב"א א"ד הם ידועים | |
|
ולפי שזוית גב"ד שוה לזוית בט"א | |
|
אבל גם זוית בג"ד הנצבת שוה לזוית אה"ט הנצבת | |
|
אם כן [כיחס ד"ג אל ג"ב כן][151] יחס א"ה אל ה"ט | |
|
ויחס ג"ד אל ג"ב ידוע א"כ גם יחס א"ה אל ה"ט ידוע | |
|
וא"ה ידוע אם כן גם ה"ט ידוע | |
|
והם מקיפים בזוית נצבת אם כן גם א"ט ידוע | |
|
ולפי שכל אחת מב"ה ה"ט ידוע אם כן גם השטח נצב הזויות ידוע אשר יקיפו בו בט"ה ויהיה שוה לשטח אשר יקיפו בו אט"ח | |
|
|
והזוית אשר אצל כל אחת מה' ח' נצבת | |
|
אם כן גם ח"ט ידוע ולכן יהיה גם א"ח ידוע | |
|
אבל גם ח"ז שוה לב"ג אם כן גם א"ז כולו יהיה ידוע | |
|
ויהיה אופן המעשה כן | |
|
אם צלע א"ב יהיה י"ג אמו' ואם צלע ב"ג י' אמות ואם צלע ג"ד כ' אמות ואם צלע ד"א י"ז אמו' [152]ונרצה למצוא התשבורת הנז' | |
|
ויהיה אם עמוד א"ה בכח צ"ו וחצי [וחמישית וחלק][153] מט"ו | |
|
ואם ב"ה יהיה בכח ע"ב [וחמישית][154] | |
|
ואם ב"ד יהיה בכח ת"ק | |
|
ולפי שאם צלע ג"ד היא כ' אמות ואם ג"ב היא עשר אמות א"כ מרובעם יהיה ת' אמות וק' אמות ועשה כך | |
|
ראה כיחס ת' אל הק' מה היחס אל צ"ו ורביעית וחמישית | |
|
ותמצא שהוא אל כ"ד וחמישית וכן מרבע ה"ט | |
|
וכפל הע"ב על כ"ד וחמישית וקח גדר ההוה וכפלהו בשנים וההוה הוא ככפל השטח הנצב הזוית אשר יקיפו בו ב"ה [ה"ט][155] | |
|
ונוסיף מרובע ב"ה [ה"ט][156] כלומר מרובע ע"ב וחמישית וכ"ד וחמישית מחוברים | |
|
ונדע ב"ט בכח שהוא ק"פ | |
|
וחבר הצ"ו וחצי וחמישית ועשירית והכ"ד וחמישיתו ויעלה קכ"ד | |
|
וכפל הק"ף על הכ"ד וחמישית יהיה בכח ד' אלפים שנ"ו | |
|
וחלקם על הקכ"א יהיה ל"ו | |
|
ונגרע מקכ"א בכח ל"ו בכח וישארו כ"ה בכח שהם ה' באורך | |
|
ותוסיף כל שעור ב"ג והוא י' אמות וההוה ט"ו וזהו שעור העמוד | |
|
ואם ה"ט בכח כ"ד וחמישית ואם ח"ט באורך ו' ואם [א"ט][157] באורך י"א | |
|
ואם תרצה למדוד נוטה אבג"ד אשר הזוית שאצל ג' נצבת ויהיה צלע א"ב י"ג אמות וצלע ב"ג י' אמות וצלע ג"ד ח' אמות וצלע א"ד כ"ה אמות כזה ותרצה למצוא תשברתו | |
|
תעשה כן | |
|
תכפול הי' על עצמם יעלו ק' | |
|
גם הח' על עצמם יעלו ס"ד | |
|
תחברם יעלו קס"ד | |
|
גם תכפול הי"ג על עצמם יעלו קס"ט | |
|
חברם עמם יעלו של"ג | |
|
גם כפול הכ"ה על עצמם יעלו תרכ"ה | |
|
גרע של"ג ישארו רצ"ב | |
|
קח חציים והם קמ"ו | |
|
כפלם על עצמם יעלו כ"א אלף שי"ו | |
|
חלקם על קס"ד יהיה החלק קכ"ט וק"ס חלקים מקס"ד באחד | |
|
גרעם מן קס"ט ישארו ל"ט וד' חלקים מן קס"ד באחד | |
|
| ||
|
כפלם על קס"ד יעלו ו' אלפים ות' | |
|
וגדרו פ' | |
|
וחציים מ' ויהיה תשבורת משולש אב"ד מ' אמות | |
|
|
||
|
אבל גם תשבורת משולש בג"ד כן מ' אמות | |
|
אם כן תשבורת כל נוטה אבג"ד פ' אמות | |
|
והמופת על המדידה הזאת | |
|
נגיע ב"ד | |
|
ויהיה תשבורת משולש בג"ד ידוע | |
|
ומרובע ב"ד יהיה קס"ד אמות | |
|
אבל גם מרובע א"ב קס"ט אמות | |
|
א"כ מרובע א"ב ב"ד [הם][158] של"ג אמות והם פחותים ממרובע א"ד | |
|
אם כן זוית אב"ד נרוחת | |
|
ואוציא עמוד א"ה על ד"ב בהוציאי אותו עד ה' | |
|
א"כ מרובע א"ד יותר גדול ממרובעי א"ב ב"ד ככפל השטח הנצב הזויו' אשר יקיפו בו [ב"ד ב"ה | |
|
אם כן כפל השטח הנצב הזויות אשר יקיפו בו][159] קוי [ב"ד][160] ב"ה ידוע | |
|
לכן גם השטח הנצב הזויות אשר יקיפו בו ב"ד ב"ה יהיה ידוע ויהיה גדר מרובע ב"ד על [מרובע][161] ב"ה | |
|
א"כ מרובע ב"ד על מרובע ב"ה ידוע | |
|
אבל גם מרובע ב"ד [אם כן][162] גם מרובע ה"ב הנשאר ידוע | |
|
|
והזוית אשר אצל ה' נצבת | |
|
א"כ גם מרובע א"ה ידוע | |
|
לכן גם מרובע א"ה על מרובע ב"ד ידוע | |
|
ויהיה גדרו השטח הנצב הזויות אשר יקיפו בו א"ה ב"ד אם כן גם שטח הנצב הזוית אשר יקיפו בו א"ה ב"ד ידוע | |
|
וחציו הוא משלש אב"ד שהוא מ' אמות כמו שזכרנו | |
|
[163]אם כן גם משלש אב"ד ידוע לכך גם כל בעל ד' צלעות אבג"ד יהיה ידוע | |
Chapter Five on Knowing the Round Shapes |
הפרק החמישי בידיעת התמונות העגולות | |
| Know that the circle is a line, within which there is a point, such that all the lines drawn from it to the circumference are equal. | דע שהעגולה היא קו אחד בתוכה נקדה שכל הקוי' היוצאים ממנה אל המקיף שוים | |
| This chapter includes the measurement of the whole circle that we have defined, as well as the measurement of the segments of the circle. | והנה בזה השער יכנסו מדידת העגולה השלימה אשר גדרנוה ומדידת חתיכות העגולה | |
| The segments of the circle are of three types: | וחתיכות העגולה ג' מינים | |
|
הא' הוא חצי העגולה | |
|
השני יותר מחציה | |
|
השלישי פחות מחציה | |
| We begin with the measuring of whole circle, then we discuss the measuring of its segments. | ונחל במדידת העגולה השלימה אחר נדבר במדידת חתיכותיה | |
| Circle | ||
| We say that the area of the circle is [obtained] by that you multiply half of its diameter by half of its perimeter. | ונאמר כי תשבורת העגולה היא בשתכה את מחצית קטרה במחצית הקו המקיף אותה | |
| The ratio of its perimeter, i.e. the line surrounding the circle, to its diameter is as the ratio of 22 to 7. | והנה ערך הקו המקיף אותה ר"ל את העגולה מקטרה כערך כ"ב אל הז' | |
| We find that the ratio of the perimeter is 3⅐ according to the opinion of the geometricians that are experts in this science. | נמצא שהקו המקיף הוא בערך ג' ושביעית וזה לפי דעת חכמי המדות המדקדקים בחכמה הזאת | |
| Ptolemy relied all his calculations in the Almagest on the calculation that the diameter is a third of the perimeter, because he divided the circle into 360 degrees and the diameter into 120 degrees, and although there is no ratio between the diameter that is a straight line and the perimeter that is a circular line, the ratio exists in a number not in the magnitude. | אמנם בטלמיוס עשה כל חשבונותיו בספר המג'יסטי על חשבון שיהיה הקטר שלישית הקו המקיף כי חלק את העגולה על ש"ס מעלות והקטר על ק"כ מעלות ואעפ"י שאין יחס בין הקטר שהוא קו ישר לקו המקיף שהוא קו עגול אבל היחס יפול במספר לא בשעור | |
|
ואם רצית למצוא תשבורת העגולה מהמקיף לבד | |
|
תקח מרובע הקו המקיף וזה בשתכה אותו על עצמו וההוה תכה אותם על ז' וההוה קח מהם חלק א' מפ"ח והוא תשבורת העגולה | |
|
המשל בזה הכינו כ"ב על עצמם והם תפ"ד | |
|
כפלנום ז' פעמים והם ג' אלפים ושפ"ח | |
|
לקחנו מהם חלק מפ"ח והם ל"ח וחצי והוא תשבורת העגולה | |
|
דמיון אחר אם רצית למצוא תשבורת העגולה מהמקיף לבד | |
|
תוסיף על המקיף חציו ורביעיתו וההוה הוא תשבורת העגולה | |
|
המשל בזה הוספנו על הקו המקיף שהוא כ"ב אמות חציו ורביעיתו שהם י"ו אמות וחצי ההוה ל"ח וחצי והם תשבורת העגולה | |
|
ואם רצית תשבורת העגולה מהקטר לבד מבלתי שתביט אל הקו המקיף | |
|
כפול מרובע הקטר על י"א וחלק העולה על י"ד והעולה הוא תשבורת העגולה וזה על חשבון חכמי המדות | |
|
ובטלמיוס אמר במקום אחר כי ערך הקו המקיף אל כל האלכסון והוא ג' וח' חלקי' מששים באחד | |
|
ואין הפרש בין זה החשבון ובין חשבון חכמי המדות רק דבר מועט שאינו מזיק בחשבון לכן סמכנו את חשבוננו על דעת חכמי המדות | |
|
עוד אם רצית למצוא תשבורת העגולה מהקטר לבד | |
|
תקח הקטר וכפלהו על הקו המקיף וקח מההוה רביעיתו והוא תשבורת העגולה | |
|
המשל בזה הקטר ז' והקו המקיף כ"ב | |
|
כפלנו זה על זה וההוה קנ"ד ורביעיתו ל"ח וחצי והוא תשבורת העגולה | |
| We have already explained the method of measuring the area of the circle. | הנה כבר בארנו דרך תשבורת מדידת העגולה | |
| Segments of the circle | ||
| Now we start to explain the method of measuring its segments. | [164]ומעתה נחל לבאר דרך מדידת חתיכותיה | |
| We say that the segment of the circle is surrounded by a segment of the perimeter and by a straight line called a chord that is drawn from the end of the perimeter to its other end. | ונאמר כי [חתכת][165] העגולה היא מקפת מן קצת הקו המקיף את העגולה ומקו ישר יקרא המיתר והוא המשוך מקצה הקו המקיף אותה עד קצהו האחר | |
| When a line is drawn from half the chord to the perimeter at right angle it is called versine. | וכאשר יצא ממחצית המיתר קו על זוית נצבת אל המקיף יקרא החץ | |
| Examine each segment: | ועיין אל כל חתיכה | |
|
ואם החצי יהיה כמחצית המיתר דע שהחתיכה היא חצי עגולה | |
|
ואם יהיה קצר ממחצית היתר דע שהחתיכה היא פחותה מחצי העגולה | |
|
ואם הוא יותר ארוך ממחצית היתר החתיכה היא גדולה ממחצית העגולה | |
| Semicircle | ||
|
וכאשר היתה החתיכה חצי עגולה ורצית לדעת תשברתה | |
|
תכפול חצי היתר שהוא אלכסון החצי עם חצי הקו המקיף אותה וההוה הוא תשבורת מחצית העגולה | |
|
כי מחצית הקו המקיף העגולה שהיא החתיכה הוא רביע הקו המקיף כל העגולה השלימה | |
|
וכאשר הכית מחצית הקטר [במחצית][166] העגולה תמצא תשבורת כל העגולה | |
|
וכאשר תכה מחצית הקטר ברביע העגולה תמצא תשבורת חצי העגולה | |
|
כי כל חשבון שתכה חציו בכולו יהיה ההוה מחצית הכאת כלו [בכלו][167] | |
|
ואם תכה חציו בחציו ההוה רביע הכאת כלו בכולו | |
|
המשל בזה עגולה שלימה אשר הקו המקיף אותה כ"ב אמות ידענו שקטרה ז' אמות רצינו למצוא תשברתה | |
|
הכינו מחצית הקטר שהוא ג' וחצי על מחצית הקו המקיף שהוא י"א אמות וההוה ל"ח וחצי ידענו מזה שתשבורת העגולה ל"ח וחצי אמות | |
|
רצינו לדעת תשבורת מחצית העגולה הזאת | |
|
לקחנו מחצית הקו המקיף אותה שהוא ה' וחצי והכינו אותם עם מחצית הקטר שהוא ג' וחצי וההוה י"ט ורביע והוא תשבורת חצי העגולה | |
|
המשל בזה חצי עגולה שיהיה קטרה י"ד אמות והחץ ז' אמות | |
|
תקח מרובע הקטר והוא קצ"ו אמות ותכפלהו בי"א ההוה ב' אלפים קנ"ו | |
|
קח החלק האחד מכ"ח שהם ע"ז אמות והם תשבורת חצי העגולה | |
|
ואם רצית למצוא את תשבורתה מהחץ תעשה כך | |
|
תקח מרובע החץ ותכפלהו על י"א ותקח שביעיתו והוא תשבורת חצי העגולה | |
|
המשל בזה קח מרובע החץ שהוא ז' ומרובעו מ"ט כפלהו על י"א ההוה תקל"ט ושביעיתו ע"ז והוא תשבורת חצי העגולה | |
|
עוד חצי עגולה אשר קטרה ז' אמות וחצה חצי הקטר שהוא ג' וחצי והקו המקיף י"א אמות ותרצה לדעת תשברתה | |
|
תעשה כך | |
|
תכפול הז' אמות של הקטר עם הי"א אמות של הקו המקיף וההוה ע"ז אמות קח רביעיתם והם י"ט אמות ורביע והם תשבורת חצי העגולה | |
|
ובאופן אחר תעשה כך | |
|
תקח מרובע הקטר שהוא ז' אמות ומרובעו מ"ט כפלם על י"א וההוה תקל"ט | |
|
קח חלק א' מכ"ח והוא י"ט אמות ורביע וזהו התשבורת | |
| Segment that is less than a semicircle | ||
|
[168]רצינו למצוא תשבורת חתיכת עגולה פחות מחציה | |
|
ובזה הוא הכרח למצוא קטר כל העגולה אשר זאת החתיכה ממנה | |
|
וככה נעשה | |
|
כבר התבאר בספר היסודות לאקלידס כי כל עגולה יפלו בה שני מיתרים ויחתכו זה את זה יהיה [כפל][169] חלק המיתר בחברו האחר ככפל חלק המיתר השני בחברו | |
|
וכאשר רצינו למצוא אלכסון העגולה אשר זו החתיכה נחצבת ממנו נעבירהו חותך המיתר על זויות נצבות ויחתכהו באמצע כפי מה שהתבאר בספר היסודות לאקלידס | |
|
ונכפול חצי המיתר על מחציתו וההוה ממנו ראוי שיהיה כמו ההוה מחץ החתיכה הזאת על שארית האלכסון | |
|
וכאשר ידענו כמות אורך החץ נכפלהו על מספר שיהיה ההוה מכפלו כמו ההוה ממחצי' המיתר על מחציתו והמספר אשר כפלנו אותו עליו יחד עם החץ יהיה שעור אלכסון | |
|
המשל בזה יהיה חתיכת עגולה פחות מחציה חתיכת א"ב ויהיה מיתרה ח' אמות וחצה ב' אמות והוא ג"ד חותך על זויות נצבות את המיתר | |
|
ובהכרח שיחתוך אותו אל חצאים | |
|
וכשנוציאנו עד מקיף העגולה כאשר נשלים אותה יעבור על מרכז העגולה | |
|
והנה חתך המיתר על ד' ד' אם כן כשנעריך חצי המיתר על חציו ההוה י"ו | |
|
וכבר הנחנו החץ ב' נעריך אותו על מספר שיהיה ההוה י"ו ואין מספר כזה זולתי הח' | |
|
וידענו שהחסר מן החץ עד תשלום האלכסון הם ח' | |
|
חברנום עם החץ ביחד והיו י' והוא אורך כל האלכסון | |
|
ונעשה [כזאת הצורה][170] ונשלים העגולה | |
|
ונאמר שנוציא שני קוים מנקדת ח' שהוא חצי האלכסון ומרכז העגולה אל נקדת א' ונקדת ב' | |
|
ונכה מחצית הקטר שהוא ח"ג במחצית הקשת המקיף את החתיכה והוא קשת א"ג וההוה הוא תשבורת המשולש אשר שתי צלעותיו ח"א ח"ב ותושבתו קשת אב"ג | |
|
אחר כך נכה מחצית המיתר בעמוד המשולש הוא קו [ח"ד][171] וההוה הוא תשבורת המשולש ישר הקוים | |
|
נגרע התשבורת [הזאת][172] מתשבורת המשולש אשר תושבותיו קשת אב"ג ואשר ישאר הוא תשבורת החתיכה הדרושה | |
|
וכאשר רצינו לדעת זאת המדידה באופן אחר מבלתי שנצטרך להוציא כל אלכסון העגולה | |
|
נחבר המיתר והחץ ונקח חציים ונכה אותם עם החץ גם נכה חצי המיתר על עצמו ונקח מהכאת חצי המיתר על עצמו חלק א' מן ארבעה עשר ונוסיף אותם על הכאת חצי המיתר והחצי עם החץ והוא התשבורת | |
|
כגון מיתר י"ב עם חץ ד' שהם י"ו וחציים ח' | |
|
נכה בד' הם ל"ב | |
|
וחצי המיתר על עצמו הם ל"ו חלקו מי"ד ב' אמות וחצי וחלק מי"ד | |
|
נוסיפם [173]על הל"ב אמות יהיה תשבורת החתיכה ל"ד אמות וחצי וחלק א' מי"ד | |
|
| ||
| Segment that is greater than a semicircle | ||
|
נרצה למצוא תשבורת חתיכת העגולה אשר היא גדולה מחציה | |
|
והחתיכה הזאת היא קשת אב"ג עם מיתר א"ג | |
|
ותוכל לדעת התשבורת כשתמצא קטר העגולה אשר זאת החתיכה היא חתיכה ממנה | |
|
ותהיה כופל חצי הקטר בחצי הקשת ותהיה מוסיף עליו תשבורת המשולש אשר תושבתו המיתר וראשו מרכז העגולה וההוה הוא תשבורת החתיכה | |
|
ונעמוד על ידיעת האלכסון כן | |
|
נקח מרובע חצי המיתר ונחלקהו על החץ ונוסיף העולה על החץ וההוה הוא אלכסון העגולה אשר זאת החתיכה נחתכה ממנה | |
|
המשל בזה יהיה חתיכת העגולה קשת אב"ג והמיתר א"ג וארכו י"ב אמות והחץ ב"ד וארכו ח' אמות | |
|
לקחנו מרובע חצי היתר שהוא ל"ו וחלקנוהו על החץ שהוא ח' ועלה החלק ד' אמות וחצי | |
|
הוספנום על החץ והם י"ב אמות וחצי וידענו שהאלכסון הוא י"ב אמות וחצי | |
|
רצינו למצוא את התשבורת | |
|
ולקחנו חצי הקטר שהוא ו' אמות ורביע וכפלנו אותו על חצי הקשת וההוה שמרנוהו | |
|
אחר כך מצאנו תשבורת המשולש [שתושבתו][174] א"ג ועמודו ה"ד ושתי צלעותיו הם א"ה ג"ה | |
|
ומצאנוהו כן ידענו שאורך כל א' מצלעותיו השתים הוא כארך חצי הקטר ולכן יהיה מרובע הצלע כמרובע העמוד ומרובע מחצי' המיתר כי הוא תושבת לזוית הנצבת | |
|
ואחרי שמרובע מחצית המיתר ל"ו נגרעהו ממרובע הצלע שהוא ל"ט אמות וחלק א' מי"ו נשארו ג' אמות וחלק א' מי"ו והם מרובע ארך העמוד | |
|
כפלנו העמוד עם מחצי' התושבת שהם ו' אמות וההוה הם י"ח אמות ושלישית וחלק מל' גם אחד מק"כ והוא תשבורת משלש אה"ג | |
|
הוספנו על השמור וההוה הוא תשבורת החתיכה הנזכרת | |
|
ואם רצינו למצוא התשבורת הזאת מבלי שנצטרך להוציא האלכסון נעשה כך | |
|
נכה המיתר עם החץ וההוה נכה אותו עם י"א ומן ההוה נקח חלק א' מי"ד והוא תשבורת החתיכה הזאת | |
|
המשל בזה חתיכת עגולה גדולה מחציה היא קשת אב"ג ומיתרה א"ג וארכו כ"ד אמות והחץ י"ו אמות | |
|
הכינו החץ עם המיתר ההוה שפ"ד אמות | |
|
הכינו שפ"ד עם י"א ההוה ד' אלפים ורכ"ד | |
|
לקחנו מהם חלק א' מהי"ד וההוה ש"א אמות וחצי ושביעית וחלק מי"ד והוא תשבורת החתיכה הנזכרת | |
| Finding the arc | ||
| Said Mordecai: since for these measurements we need to find the size of the arc surrounding the segments of the circle and this is explained in the tables of the chords and arcs, because by knowing the chord we know the arc and by knowing the arc we know the chord, as Ptolemy explained in the first book of the Almagest, and writing tables is not the way of our book, I saw fit to show you a way to know the arcs without having to use these tables. | אמר מרדכי בעבור שאלה המדידות אנו צריכים למצוא כמות הקשת המקפת בחתיכו' העגולה וזה מתבאר בלוחות המיתרים והקשתות כי בידיעת המיתר נדע הקשת גם בידיעת הקשת נדע המיתר כמו שביאר זה בטלמיוס במאמר א' מספר האלמג'יסטי [175]מאג'יסטי ואין מדרך חבורנו זה לכתוב בו לוחות ראיתי להמציא לך דרך לדעת הקשתות מבלי שתצטרך אל הלוחות ההם | |
|
ואומר אם חתכת העגולה תהיה פחות מחציה ותרצה לדעת כמות הקשת המקיף אותה תעשה כך | |
|
לעולם תחבר המיתר על החץ ותגרע מהם רביעיתם וקח מהנשארים רביעיתם והוסף עליהם וההוה הוא כמות הקשת המקיף את החתיכה | |
|
המשל בזה תהיה חתיכת עגולה פחות מחציה חתיכת א"ב ומיתרה מ' אמות וחצה י' אמות | |
|
חברנו המיתר עם החץ ההוה נ' אמות | |
|
גרענו אלו י"ב אמות וחצי שהם רביעיתם נשארו ל"ז אמות וחצי | |
|
לקחנו ט' אמות ורביע ושמינית שהם רביע הל"ז אמות וחצי אשר נשארו | |
|
והוספנו אותם עליהם ההוה מ"ו אמות וג' רביעיות ושמינית והוא כמות הקו המקיף את החתיכה | |
|
| ||
|
אמנם גרענו רביע והוספנו רביע בעבור שהחץ הוא רביעית חלק מהמיתר | |
| Shapes with curved sides | ||
| Having explained all of these, it is now possible to measure all shapes with a curved side. | ואחר שבארנו [כל אלה][176] מעתה אתה יכול למדוד כל הצורות שיש להם צלע עקום | |
|
כגון משלש אב"ג אשר שתי צלעותיו ישרים ותושבתה קשת עגול והוא קשת ב"ג | |
|
שתמדוד המשלש בפני עצמו וזה בשתמשוך קו ישר מב' עד ג' | |
|
ותוציא העמוד על קו ב"ג | |
|
ותכהו במחצית התושבת כמו במדידת המשלשים | |
|
עוד נמצא את הקשת הזה במצאינו עגולה מאיזו נחצבה כמו שבארנו במדידת החתיכות שהם פחותות מחצי עגולה או עודפות על חצי עגולה | |
|
ונמצא תשבורתה ונחבר אותה עם תשבורת המשולש והמחובר הוא תשבורת התמונה הזאת | |
| We do so also when there are two curved sides and the other is a straight line, like this: | גם ככה נעשה בהיות שתי הצלעות עקומו' כזה והאחרת קו ישר | |
|
כגון תמונת אב"ג | |
|
שנמשך שני קוים ישרים מנקודת א' וב' אל נקדת ג' | |
|
ונעשה משלש ישר הקוים ונמדוד אותו כמשפט | |
|
עוד נמדוד שני העקומות כל אחת בהיותה חתיכת עגולה כי שתי צלעות המשולש הם מיתרים לשתי החתיכות הנזכרות | |
|
ונחבר שלשת המדידות כלומר מדידת המשולש ומדידת כל אחת משתי החתיכות וההוה הוא תשבורת תמונה הזאת | |
|
גם ככה אם היו שתי צלעותיה עקומות ובם תשלם התמונה כמו תמונת אב"ג | |
|
שנוציא קו ישר מנקדת ב' אל נקדת ד' ויהיה מיתר לשתי חתיכות של העגולה לחתיכת בא"ד ולחתיכת בג"ד | |
|
ונמדוד כל חתיכה בפני עצמה ונחבר שתי המדידות והם יהיו תשבורת התמונה | |
Chapter Six on the Measuring of Regular Polygons |
הפרק הששי במדידת התמונות רבות הזויות | |
| know that every polygon is divided into triangles, even the triangle itself. | דע שכל תמונה ישרת הקוים תחלק אל משלשים ואפי' המשולש עצמו | |
| As explained in the books of the ancients that the triangle is a root and a foundation for all the polygons, because they are formed from it and decomposed to it. | כמו שהתבאר בספרי הקדמונים שהמשולש הוא שרש ויסוד לכל התמונות ישרות הקוים [177]כי מהם התהוו ואליהם יותכו | |
| You already know the measurement of the various triangles. | וכבר ידעת מדידת המשולשים למיניהם | |
| When you want to measure a polygon, draw a straight line from each of its vertices to the center of the shape, then divide it into triangles as the number of the vertices. | וכאשר תרצה למדוד תמונה רבת הזויות תוציא קו ישר מכל זוית מזויותיו אל מרכז התמונה ואז תחלק למשלשי' בכמות הזויות | |
| When you measure all the triangles and sum their values, the sum is the area of this shape. | וכאשר תמדוד כל המשלשים ותקבץ מנינם המקובץ הוא תשבורת התמונה ההיא | |
| If the sides of the triangles are equal, i.e. their bases, and so are their legs, so that you can draw a circle, [whose center is] the center of the shape, that touches each of its vertices, you measure one triangle alone and find its area. | ואם צלעות המשולש שוים כלומר תושבותיהם גם השוקים שלהם וזה כשתעגל ממרכז התמונה ובמרחק זוית מזויותיה עגולה ותמשש כל הזויות אז תמדוד המשלש הא' לבד ותמצא תשברתו | |
| Then you multiply the area mentioned by the number of the vertices and the result is the area of this shape. | אח"כ תכה את התשבורת הנזכרת במנין הזויות וההוה הוא תשבורת התמונה | |
| This is the rule that is given for the measurement of the polygons. | וזהו הכלל הנתון במדידת התמונות רבות הזויות | |
| In order to make it easier for the one who calculates, the scholars gave [other] ways to measure them, so we will write them here. | אמנם כדי להקל על המחשב נתנו החכמים דרכים במדידתם ולכן נכתבם פה | |
| We start by measuring the pentagon [lit. a shape that has five sides and five vertices], because we have already mentioned the triangles and the rectangles. | ונחל ממדידת תמונה בעלת ה' צלעות ובעלת ה' זויות כי המשלשים גם המרובעים כבר זכרנום | |
| The shapes that we will mention are those that the circle touches their vertices [= cyclic polygons] and that are equilateral and equiangular [= regular polygons]. | ואלה התמונות אשר נזכיר הם שתמשש העגולה זויותיהם ותהיינה שוה הצלעות והזויות | |
| We say: | ונאמר | |
| Pentagon | ||
|
אם רצית למדוד תמונה בעלת חמש זויות | |
|
תעשה ככה | |
|
תכפול הצלע על עצמו וההוה כפלהו ה' פעמים וקח שלישיתו והוא תשבורת התמונה הנזכרת | |
|
המשל יש לנו תמונת אבגד"ה בעלת ה' זויות וכל צלע מצלעותיה י' אמות | |
|
כפלנו הי' על עצמו וההוה ק' | |
|
כפלנו זה על ה' וההוה ת"ק | |
|
לקחנו שלישיתם והם קס"ו אמות וב' שלישי אמה והם תשבורת התמונה | |
|
ואם תרצה למצוא גם קטר העגולה הממששת התמונה תכפול הצלע שהם עשרה אמות על י"ז ההוה ק"ע חלקם על י' וההוה י"ז והם קטר העגולה | |
| Hexagon | ||
|
ואם רצית למדוד תמונת בעלת שש צלעות ושש זויות | |
|
תעשה כך | |
|
תכפול הצלע על עצמו וההוה כפלהו על ו' ותקח מהם השלישית והעשירית והם תשבורת התמונה | |
|
המשל יש לנו תמונת אבגדה"ו וכל צלעותיה ל' אמות והקטר ס' אמות | |
|
כפלנו הל' על עצמו וההוה תת"ק | |
|
כפלנום על ו' ההוה ה' אלפים וארבע מאות | |
|
לקחנו מהם השלישית והעשירית ההוה ב' אלפים ש"מ והוא תשבורת התמונה | |
|
| ||
| Heptagon | ||
|
ואם רצית למדוד תמונה בעלת שבע זויות | |
|
תעשה כך | |
|
תכפול הצלע על עצמו וההוה כפול אותו על מ"ג ומההוה קח חלק א' מי"ב וההוה הוא תשבורת התמונה | |
|
המשל יש לנו תמונת אבגדהו"ז וכל צלע מצלעותיה י' אמות | |
|
כפול הי' על עצמם ההוה כפלם על מ"ג ההוה ד' אלפים ש' | |
|
קח חלק [178]אחד מי"ב וההוה שנ"ח ושליש והם תשבורת התמונה | |
| Octagon | ||
|
ואם רצית למדוד תמונה בעלת שמנה זויות | |
|
תעשה כך | |
|
תכפול הצלע על עצמו וההוה כפלהו על כ"ט וקח ששיתו והוא תשבורת התמונה | |
|
המשל יש לנו תמונת אבגדהוז"ח וכל צלע מצלעותיה י' אמות | |
|
כפלנו הי' על עצמם העולה ק' | |
|
כפלנו הק' על כ"ט ההוה ב' אלפים תת"ק | |
|
לקחנו את ששיתו ההוה תפ"ג ושליש והוא תשבורת התמונה | |
| Nonagon | ||
|
ואם רצית למדוד תמונה בעלת ט' צלעות וט' זויות | |
|
תעשה כך | |
|
תכפול הצלע על עצמו וההוה כפלהו על נ"א וקח שמיניתם והוא תשבורת התמונה | |
|
המשל יש לנו תמונת אבגדהוזח"ט וכל צלע מצלעותיה י' אמות | |
|
כפלנו העשרה על עצמם ההוה ק' | |
|
כפלנום על נ"א וההוה ה' אלפים ק' | |
|
לקחנו שמיניתם והם תרל"ז אמות וחצי וככה תשבורת התמונה | |
| Decagon | ||
|
ואם רצית למדוד תמונה בעלת י' צלעות וי' זויות | |
|
תעשה כך | |
|
תכפול הצלע על עצמו וההוה קח אותו וכפלהו על ט"ו וקח חצי ההוה והוא תשבורת התמונה | |
|
והמשל בזה יש לנו תמונת אבגדהוזחט"י וכל צלע מצלעותיה י' אמות | |
|
כפלנו הי' על עצמם והיו ק' | |
|
כפלנום על ט"ו ההוה אלף ת"ק | |
|
וחציים שבע מאות וחמשי' וככה תשבורת התמונה | |
|
ובפנים האחרים גם כן | |
|
כפלנו הי' על עצמם וההוה ק' | |
|
כפלנום על ל"ח וההוה ג' אלפים ות"ת | |
|
לקחנו חמישיתו והם תש"ס והוא תשבורת התמונה וזה החשבון יותר מדוקדק | |
| Hendecagon | ||
|
ואם רצית למדוד תמונה בעלת י"א זויות | |
|
עשה כך | |
|
תכפול הצלע על עצמו וההוה כפלהו על ס"ו ומן ההוה קח שביעיתו והוא תשבורת התמונה | |
|
המשל יש לנו תמונת אבגדהוזחטי י"א וכל צלע מצלעותיה י' אמות | |
|
כפלנו הצלע על עצמו ההוה ק' | |
|
כפלנום על ס"ו והיו ו' אלפים ת"ר | |
|
לקחנו שביעיתם והם תתקמ"ג וככה תשבורת התמונה | |
| Dodecagon | ||
|
ואם רצית למדוד תמונה בעלת י"ב זויות | |
|
תעשה כך | |
|
תכפול הצלע על [179]עצמו וההוה כפלהו על מ"ה ומההוה קח רביעיתם וככה תשבורת התמונה | |
|
המשל יש לנו תמונת א'ב'ג'ד'ה'ו'ז'ח'ט'י' י"א י"ב וכל צלע מצלעותיה י' אמות | |
|
כפלנו הצלע על עצמו היו ק' | |
|
כפלנום על מ"ה וההוה ד' אלפים ת"ק | |
|
לקחנו רביעיתם והם אלף קכ"ה וככה תשברתם | |
| The other polygons that are not equilateral and equiangular are measured by measuring the triangles into which they are divided. | ושאר התמונות רבות הזויות אשר אינן שוות הצלעות והזויות נמדדות במדידת המשולשים אשר עליהן תחלקנה | |
| Said Mordecai: I have taught you the measurement of the polygons from the pentagon to the dodecagon easily and without effort. | אמר מרדכי הנה הודעתיך מדידת התמונות רבות הזויות מן המחומש עד בעל י"ב זויות בקלות ובלי יגיעה | |
| I did not bring proof to every shape so as not to confuse the student, I only brought the procedure. | אמנם לא הבאתי המופת על כל תמונה ותמונה כדי שלא אבלבל התלמיד אבל הבאתי אופן המעשה [בלבד | |
| Now, I will bring a proof for the measurement of each shape and start with the pentagon, as I started the procedure. | ועתה אביא המופת בעבור כל מדידת תמונה ותמונה ואחל מהמחומש][180] כמו שהחלותי באופן המעשה | |
| Before I begin with the proof I will mention two triangles that will help to us in what we want to explain: | [וטרם שאחל להביא המופת אקדים להזכיר][181] שני משולשים להיותם לעזר במה שנרצה לבאר | |
| Equilateral triangle | ||
|
ונאמר משולש אב"ג שוה הצלעות אשר כל אחת מצלעותיו י' אמות | |
|
אוציא עמוד א"ד על קו ג"ב | |
|
לפי שקו ב"ג כלו' קו ב"א הוא כפל ב"ד אם כן מרובע א"ב הוא ד' כפלי מרובע ב"ד | |
|
ולכן מרובע א"ד הוא שלשה כפלי מרובע ד"ב | |
|
|
ומרובע ג"ב ד' כפלי מרובע ד"ב | |
|
א"כ מרובע ב"ג הוא חלק א' ושליש ממרובע א"ד | |
|
אם כן יחס מרובע ב"ג אל מרובע א"ד כיחס ד' אל ג' | |
|
ולעולם על מרובע ב"ג כלו' שיכפל [מרובע] ב"ג על עצמו ומרובע א"ד על מרבע ב"ג אם כן כח מרובע ב"ג אצל מרובע ב"ג על מרובע א"ד יחסו כיחס ד' אל ג' שהוא כיחס י"ו אל י"ב | |
|
| ||
|
ומרובע ב"ג על מרובע א"ד הוא ככפל השטח הנצב הזויות אשר יקיפו בו א"ד ב"ג על עצמו כלומר שני משולשים על עצמם | |
|
אם כן כחו של מרובע ב"ג על כפל שני משלשים על עצמם יחסם כיחס י"ו אל י"ב | |
|
וכפל שני משלשים על עצמם הוא ד' כפלי משלש א' על עצמו | |
|
[אם כן כחו על מרבע ב"ג אצל כפל משלש אחד על עצמו יחסו כיחס י"ו אל ג'] | |
|
אם כן כחו של מרובע ב"ג ידוע [אם כן גם תשברת המשלש על עצמו ידוע] ולכן גם המשולש עצמו ידוע | |
|
ואופן המעשה כן | |
|
כפול הי' על עצמם יעלו ק' | |
|
עוד הק' על עצמם יעלו רבבה אחת | |
|
קח מאלה שלישית מחציתם וחלק א' מכ"ד יהיה אלף תתע"ה | |
|
קח גדרם ולפי שאין להם גדר [מדבר] אני לוקח גדרו הקרוב ויהיה התשבורת מ"ג ושליש וחלק מל"ח וחלק ממ' וחלק ממ"א | |
|
| ||
| Right-angled triangle | ||
|
אם תרצה לדעת משלש נצב הזויות והוא משלש אב"ג | |
|
והזוית אשר אצל ג' נצבת | |
|
ואשר אצל א' שני חומשי נצבת | |
|
ונאמר כי המרובע ההוה משני קוי ב"א א"ג ביחד הוא כפלי ה' פעמים מרובע א"ג | |
|
אוציא א"ג עד ד' | |
|
ואשים גם א"ג שוה לג"ד | |
|
ואגיע ב"ד | |
|
א"כ אם א"ב שוה לב"ד | |
|
|
ואם זוית אב"ג שוה לזוית גב"ד | |
|
אבל זוית גב"ד היא ג' חומשי נצבת | |
|
בעבור שזוית בא"ג היא שני חומשי נצבת | |
|
אם כן זוית אב"ד היא ו' חומשי נצבת | |
|
א"כ זוית אב"ד [182]היא זוית של בעלת ה' זויות | |
|
|
וא"ב שוה לב"ד | |
|
א"כ קו א"ד נחלק על יחס אמצעי ושני קצוות והחלק הגדול הוא קו א"ב | |
|
|
וקו א"ג הוא חצי א"ד | |
|
אם כן המרובע ההוה משני קוי ב"א א"ג הוא [חמשה] כפלים ממרובע א"ג | |
| Pentagon | ||
|
ואומר אחוק מחומש אבגד"ה אשר כל אחד מצלעותיו י' אמות וארצה למצוא תשברתו | |
|
אקח מרכז העגולה המקפת את התמונה והיא ז' | |
|
ואגיע ז"ג ז"ד | |
|
ואעמיד עמוד ז"ח על קו ג"ד | |
|
אם כן זוית גז"ד היא ד' חומשי הנצבת | |
|
אם כן זוית גז"ח היא ב' חומשי הנצבת | |
|
והזוית אשר יקיפוה קוי ג"ח ח"ז היא הנצבת | |
|
אם כן המרובע ההוה משני קוי ג"ז ז"ח מדובקים הם ה' כפלי מרובע ז"ח | |
|
אבל לפי שבמספרים לא יתכן למצוא ה' כפלי מרובע שיהיה מרובע ראוי שנקח הקרוב לו ויהיה הפ"א אל י"ו | |
|
אם כן יחס קו ג"ז ז"ח מדובקים אל קו ז"ח כיחס ט' אל ד' | |
|
וכשנבדיל יהיה יחס קו ג"ז אל ז"ח כיחס ה' אל ד' | |
|
אם כן יחס מרובע ג"ז אל מרובע ז"ח כיחס כ"ה אל י"ו | |
|
גם הנשאר מרובע ג"ח אל מרובע ז"ח כיחס ט' אל י"ו | |
|
אם כן יחס ג"ח אל ח"ז כיחס ג' אל ד' | |
|
לכן יחס ג"ד אל ז"ח כיחס ו' אל ד' שהוא כיחס ג' אל ב' | |
|
א"כ יחס מרובע ג"ד אל השטח אשר יקיפו בו קוי ג"ד ז"ח כיחס ג' אל ב' | |
|
ויהיה מרובע ג"ד ידוע א"כ יודע גם השטח המוקף מקוי ג"ד ז"ח והוא כפל משלש גז"ד והוא חלק חמישי מתמונת אבגד"ה בעלת חמשת זויות | |
|
יהיה אם כן גם תמונת אבגד"ה ידוע ויהיה תשברתו קס"ו אמות וב' שלישי אמה בקרוב | |
|
ואם ימצא מרובע אחר ויהיה יותר קרוב לה' כפלי מרובע אחר אז נמצא תשבורת התמונה מדוקדקת יותר | |
| Hexagon | ||
| I shall further declare the proof of the hexagon and say: | עוד אודיע מופת המשושה ואומר | |
|
אחוק משושה עליו אבגדה"ו אשר כל אחת מצלעותיו י' אמות וארצה למצוא תשברתו | |
|
אקח מרכז העגולה המקפת אותו והוא ח' | |
|
ונגיע ג"ח ח"ד | |
|
א"כ ג"ד שוה לכל אחד מן ג"ח ח"ד | |
|
אם כן משולש גח"ד שוה הצלעות וצלעו ידועה | |
|
אם כן גם משולש גח"ד ידוע והוא ששית חלק המשושה | |
|
אם כן גם משושה אבגדה"ו ידוע | |
| Since the method of the procedure is different than what we have written at first, I shall write it here: | ובעבור שאופן המעשה בזה על פנים אחרים ממה שכתבנו תחלה אכתבנו פה | |
|
נכפול הי' על עצמם ההוה ק' | |
|
עוד הק' על עצמם הוא רבבה | |
|
ונקח רביעיתם והם אלפים ב' ות"ק | |
|
כפלם עם כ"ז ההוה ס"ז אלפי' ות"ק | |
|
קח שרשם והם רנ"ט וככה תשבורת משושה | |
|
|
||
Proposition: if you draw a hexagon in a circle, the ratio of the line that is drawn from the center of the circle to the side of the hexagon is 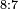
|
הקדמה אם תחקק בעגולה תמונה בעלת ו' צלעות שוות וז' זויות הקו אשר יצא ממרכז העגולה יחסו אל צלע התמונה בעלת ז' הזויות יחס הח' אל הז' | |
|
ויהיה עגולת ב"ג על מרכז א' | |
|
ואחוק עליו צלע התמונה בעלת שש הזויות והוא צלע ב"ג כלו' שתהיה שוה לקו היוצא ממרכז העגולה | |
|
ואעמיד עליה העמוד א"ד | |
|
אם כן יהיה א"ד בקרוב שוה [183]לצלע בעלת ז' זויות | |
|
ונגיע קוי ב"א א"ג | |
|
אם כן משלש אב"ג שוה הצלעות | |
|
אם כן מרובע א"ד הוא ג' כפלי מרבע ד"ב | |
|
|
א"כ יחס ד"א אל ד"ב בכח כיחס מ"ט אל י"ו בקירוב | |
|
|
ובאורך יחס ד"א אל א"ב כיחס ז' אל ד' | |
|
|
ויהיה ב"ג כפל ב"ד | |
|
אם כן יחס ב"ג אל ד"א כיחס ח' אל ז' | |
| Heptagon | ||
|
ואם תרצה למדוד תמונה בעלת ז' זויות | |
|
אחוק תמונת אבגדהו"ז אשר כל צלעותיו בעלי י' אמות ותרצה למצוא תשברתה | |
|
אקח מרכז העגולה המקפת אותה והיא נקדת ט' | |
|
ואגיע קו ד"ט ט"ה | |
|
ואעמיד עמוד ט"ד על קו ד"ה | |
|
אם כן יחס ט"ד אל ד"ה כיחס ח' אל ג' | |
|
ואל ד"כ כיחס ח' אל ג' וחצי והוא כיחס י"ו אל ז' | |
|
לכן יחס ט"כ אל כ"ד כיחס י"ד ושליש אל ז' בקרוב והוא יחס מ"ג אל כ"א | |
|
לכן גם יחס ד"ה אל כ"ט כיחס מ"ב אל מ"ג והוא יחס פ"ד אל פ"ו | |
|
אם כן מרובע ד"ה אצל השטח הנצב הזויות אשר יקיפו בו ד"ה כ"ט הוא כזה היחס בעצמו | |
|
אם כן יחסו אל משלש דה"ט כיחס פ"ד אל מ"ג | |
|
ויחס המשלש אל בעלת ז' זויות כיחס א' אל ז' | |
|
א"כ יחסו של מרובע ד"ה אל בעלת ז' זויות כיחס י"ב אל מ"ג | |
|
ומרובע ד"ה ידוע אם כן גם בעלת ז' זויות ידועה | |
| Octagon | ||
|
ואם תרצה למדוד תמונה בעלת ח' זויות | |
|
אחוק תמונת אבגדהוז"ח אשר כל צלע מצלעותיה בעלת י' אמות ותרצה למצוא תשברתה | |
|
אקח מרכז העגולה המקפת אותה והיא נקדת כ' | |
|
ואגיע קוי ד"כ ה"כ | |
|
ואעמיד עמוד כ"ל על קו ד"ה | |
|
אם כן זויות דכ"ה היא חצי נצבת | |
|
לכן היא זוית דכ"ל רביע נצבת | |
|
ואעשה זוית כד"מ שוה לה | |
|
אם כן גם זוית כד"מ רביע נצבת | |
|
אם כן זוית דמ"ל חצי נצבת | |
|
והזוית אשר אצל ל' נצבת | |
|
אם כן קו ד"ל שוה לקו מ"ל | |
|
אם כן מרובע ד"מ כפל מרובע מ"ל | |
|
אם כן יחס ד"מ אל מ"ל כיחס י"ז אל י"ב בקרוב | |
|
|
וד"מ שוה למ"כ | |
|
אם כן יחס כ"מ אל מ"ל כיחס י"ז אל י"ב | |
|
אם כן יחס כ"ל אל מ"ל שהוא ד"ל כיחס כ"ט אל י"ב | |
|
אם כן יחסו אל ד"ה כיחס כ"ט אל כ"ד | |
|
אם כן יחס מרובע ד"ה אל שטח נצבת הזויות אשר יקיפו בו קוי ד"ה כ"ל כיחס כ"ד אל כ"ט | |
|
אם כן יחסו אל משלש כה"ד כיחס כ"ד אל י"ד וחצי | |
|
ויחסו א"כ אל תמונת אבגדהוז"ח בעלת ח' זויות כיחס כ"ד אל קי"ו שהם כיחס ו' אל כ"ט | |
|
ומרובע ד"ה ידוע אם כן גם התמונה בעלת ח' זויות ידועה | |
| Nonagon | ||
|
ואם רצית למדוד תמונה בעלת ט' צלעות וט' זויות | |
|
אחוק תמונת אבגדהוזח"ט ויהיה כל צלע מצלעותיה בעל י' אמות ותרצה למצוא תשברתה | |
|
אחוק עגולה סביב התמונה | |
|
ויהיה מרכזה נקדת ל' | |
|
ואגיע ה"ל ואוציאנה עד מ' | |
|
ואגיע מ"ז | |
|
אם כן משלש הז"מ מתמונת בעלת [184]ט' זויות ידוע | |
|
וכבר התבאר בקוי העגולה כי קו ז"ה הוא שלישית ה"מ בקרוב | |
|
אם כן מרובע ה"מ הוא ט' כפלי מרובע ה"ז | |
|
לכן מרובע מ"ז הוא ח' כפלי מרובע ה"ז | |
|
ובחצי העגולה יתחייב להיות הזויות אשר אצל ז' נצבת | |
|
אם כן יחס מרובע מ"ז אל מרובע ז"ה כיחס רפ"ט אל ל"ו בקרוב | |
|
אם כן יחס מ"ז אל ז"ה כיחס י"ז אל ו' בקרוב | |
|
לכן יחס מרובע ה"ז אל משולש המ"ז כיחס ל"ו אל נ"א כלומר כיחס י"ב אל י"ז | |
|
ואם כן יחסו אל התמונה בעלת ט' זויות כיחס י"ב אל ע"ו וחצי כלו' כיחס כ"ד אל קנ"ג שהם כיחס ח' אל נ"א | |
|
ומרובע ה"ז ידוע אם כן גם תמונת בעלת ט' זויות ידועה | |
| Decagon | ||
|
ואם רצית למדוד תמונה בעלת י' זויות | |
|
אחוק תמונת א'ב'ג'ד'ה'ו'ז'ח'ט'י' ויהיה כל צלע מצלעותיה בעל י' אמות ותרצה תשברתה | |
|
אקח מרכז העגולה המקפת אותה נקדת מ' | |
|
ואגיע מ"ה מ"ז | |
|
ואעמיד עמוד מ"נ על קו ה"ז | |
|
אם כן זוית המ"ז היא שני חומשי נצבת | |
|
לכן זוית המ"נ היא חומש נצבת | |
|
ואעמיד זוית מה"ס שוה לה | |
|
אם כן זוית נס"ה היא שני חומשי נצבת | |
|
|
וזוית הנ"ס נצבת | |
|
[אם כן] יחס ה"ס אל נ"ס כיחס ה' אל ד' | |
|
ויחסה אל ה"נ כיחס ה' אל ג' | |
|
ושוים אם ה"ס אל ס"מ ואם ה"נ אל נ"ז | |
|
ואם כן יחס ה"ז אל מ"נ כיחס ו' אל ט' שהם כיחס ב' אל ג' | |
|
וא"כ יחס מרובע ה' אל השטח נצב הזויות אשר יקיפו בו הז"מ כיחס ב' אל ג' | |
|
לכן יחסו אל משולש הז"מ כיחס ב' אל ג' | |
|
לכן יחסו אל משולש הז"מ כיחס [א'] אל א' וחצי | |
|
לכן יחסו אל התמונה בעלת י' זויות כיחס ב' אל ט"ו | |
|
ומרובע ה"ז ידוע א"כ גם התמונה בעלת י' זויות ידועה | |
| Hendecagon | ||
|
ואם רצית למדוד תמונה בעלת י"א זויות | |
|
אחוק תמונת א'ב'ג'ד'ה'ו'ז'ח'ט'י' י"א וכל צלע מצלעותיה י' אמות ותרצה למצוא תשברתה | |
|
אחוק עגולה סביב התמונה אשר מרכזה יהיה נקדת נ' | |
|
ואגיע ז"נ ואוציאנו עד ס' | |
|
ואגיע ס"ח | |
|
אם כן משולש זח"ס הם שני חלקים מי"א מתמונת בעלת י"א זויות | |
|
וכבר התבאר בקוי העגולה כי יחס ז"ס ז"ח כיחס כ"ה אל ז' בקרוב | |
|
ואם כן יחס ס"ח אל ח"ז כיחס כ"ד אל ז' | |
|
א"כ יחס מרובע ז"ח אל משולש זח"ס יחס מ"ט אל פ"ד שהוא כיחס ז' אל י"ב | |
|
ואם יחס המשולש אל התמונה בעלת י"א זויות כיחס ב' אל י"א [לכן יחס מרבע ז"ח אל התמונה בעלת אחת עשרה זויות כיחס ז'] אל ס"ו | |
|
ומרובע ז"ח ידוע אם כן גם התמונה בעלת י"א זויות ידועה | |
| Dodecagon | ||
|
ואם רצית למדוד תמונה בעלת י"ב זויות | |
|
אחוק תמונת א'ב'ג'ד'ה'ו'ז'ח'ט'י' י"א י"ב ותהיה כל צלע מצלעותיה בעלת י' אמות ותרצה למצוא תשברתה | |
|
אקח מרכז העגולה המקפת אותה והיא נקדת ס' | |
|
ואגיע ס"ח ס"ז | |
|
ואעמיד עמוד ס"ע על קו ה"ז | |
|
אם כן זוית זס"ע הוא ששית הנצבת | |
|
ואעשה זוית סז"פ שוה לה | |
|
אם כן זוית זפ"ע שלישית נצבת | |
|
אם כן מרובע פ"ע הוא שלישית כפלי מרובע ע"ז | |
|
|
אם כן יחס פ"ע אל ע"ז כיחס ז' אל ד' בקרוב | |
|
|
[185]לכן גם יחס ז"ח והוא ס"פ אל פ"ע כיחס ח' אל ז' בקרוב | |
|
|
לכן גם יחס ז"ח אל ס"ע כיחס [ח'] אל ט"ו | |
|
אם כן גם יחס מרובע ז"ח אל יחס השטח הנצב הזויות אשר יקיפו בו ז"ח ס"ע הוא כיחס [ח'] אל ט"ו | |
|
ויחסו אל משולש זח"ס יהיה א"כ כיחס ח' אל ז' וחצי | |
|
ואם כן יהיה יחסו אל התמונה בעלת י"ב זויות כיחס ח' אל צ' שהוא כיחס ד' אל מ"ה | |
|
ומרובע ז"ח ידוע אם כן גם תמונת בעלת י"ב זויות ידועה | |
[The Second Section on the Division of Plane Shapes] |
||
The First Chapter of the Second Section of the Second Book on the Division of Plane Shapes |
הפרק הראשון מהחלק השני מהספר השני בחלוקת התמונו' השטחיות | |
| We start with the triangles. | ונחל מהמשלשים | |
| If you wish to divide an isosceles triangle into two equal parts, draw a perpendicular from one of its vertices to the base, by dividing the angle into two equal parts, so the triangle is divided into two equal triangles | אם רצית לחלוק משולש שוה הצלעות לשני חלקים שוים אוציא עמוד מזוית אחד מזויותיו אל התושבת וזה בשתחלוק הזוית אל שני חלקים שוים ואז יהיה המשולש נחלק לשני משלשים שוים | |
| Do so if the triangle is equilateral triangle, draw a perpendicular from the vertex opposite to its base to the base. | וככה תעשה אם המשולש שוה ותוציא מזויתו שהיא מיתר התושבת עמוד אל התושבת | |
| Likewise, if you want to divide it into more than two parts, divide the base into as many parts you wish and draw from each part a straight line to the vertex, meaning the top of the triangle. Then the triangle is divided as the number of parts of the base. | גם אם רצית לחלקו ביותר משני חלקים תחלק התושבת בשיווי לחלקים שתרצה ותוציא מכל חלק מהם קו ישר אל הזוית כלומר אל ראש המשולש ואז יחלק המשולש בשעור חלקי התושבת | |
|
ומופת זה התבאר בתמונה הא' ממאמר ו' לאקלידס | |
|
דמיון רצינו לחלק משולש אב"ג לשני חלקים שוים | |
|
חלקנו התושבת שהוא ב"ג על נקדת ד' לשני חלקים שוים | |
|
והוצאנו עמוד מנקדת ד' אל ראש המשולש שהוא א' ובזה נחלק לשני משולשים שוים | |
|
ואם רצינו לחלקו על ג' | |
|
חלקנו התושבת על ג' חלקים שוים והם א"ד ד"ה ה"ג | |
|
אחר הוצאנו ד"א ה"א ובזה נחלק על שלשה חלקים שוים | |
| Do the same if you wish to divide it into more than that. | גם ככה תעשה אם רצית לחלקו יותר מזה | |
| If you wish to divide the triangle mentioned into two equal parts, so that one part is a triangle and the other part is a trapezium. | אמנם אם רצית לחלק המשולש הנזכר לב' חלקים שוים מהצד האחד עד שיהיה החלק משולש והאחד נוטה | |
|
כגון משולש אב"ג ונחלקהו על משולש אד"ה ועל נוטה ד"הב"ג | |
|
נעשה כך | |
|
נחלק הצלע האחד על נקדה שיהיה מרובע החלק האחד כפל מרובע החלק השני | |
|
ונוציא מהנקודה ההיא קו נכוחי לתושבת המשולש ונגיעהו על הצלע האחר | |
|
ואז נחלק המשלש לשני חלקים שוים משלש אד"ה ונוטה ד"הב"ג | |
| Euclid has already explained the proof of this in Book 6, proposition 18: that for every two similar triangles, the ratio of the triangle to the triangle is as the duplicate ratio of its side to its corresponding side. | והמופת על זה כבר ביאר אקלידס בתמונת י"ח ממאמר ו' שכל שני משלשים דומים יחס המשולש אל המשולש כיחס צלעו אל צלעו הנכחי לו שנוי | |
|
וידוע שהמשלש הגדול והוא המשולש אב"ג דומה אל המשולש הקטן והוא משולש אד"ה | |
|
כי הזוית אשר אצל ח' משותפת לשני המשולשי' | |
|
וזוית אד"ה שוה לזוית אב"ג כי הם שתי זויות המומרות אשר על קוים נכוחיים כשנפל עליהם קו ישר והחיצונה [186]שוה לשכנגדה | |
|
גם ככה זוית אה"ד שוה לזוית אג"ב לזה הענין עצמו | |
|
אם כן מיתרי הזויות השוות מתיחסות | |
|
|
יחס א"ב אל א"ד כיחס א"ה אל ה"ג וכיחס ד"ה אל ב"ג | |
|
אם כן שני המשולשים דומים | |
|
ולכן יהיה יחס משלש אב"ג אל משלש אד"ה כיחס צלע א"ב אל צלע א"ד שנוי | |
|
וזה היחס מי"ו מח' בעצמו הוא יחס צלע מרובע א"ב אל מרובע צלע א"ד | |
|
כי כאשר תכפול יחס הצלע אל הצלע בעצמו והוא אשר יקרא היחס שנוי יהיה הנולד יחס המרבע אל מרבע | |
|
דמיון אם צלע א"ב ו' אמות וצלע א"ד ב' אמות | |
|
יהיה יחס ב' אל ו' כיחס השלישית אל האחד | |
|
וכאשר תקח יחס השלישית אל האחד שנוי וזה כשתכפול השלישית על השלישית יהיה הנולד תשיעית וזהו יחס המשלש הקטן אל המשלש הגדול | |
|
גם ככה כשתקח מרובע ו' שהוא הצלע האחד הוא ל"ו ומרובע ב' שהוא צלע המשולש האחר ד' יהיה יחס ד' אל ל"ו יחס התשיעית אל האחד והוא המשלש הקטן אל הגדול | |
|
על כן כשתרצה לחלק המשולש לשני חלקים שוים נחלק צלע א"ב על נקודה והיא ד' עד שיהיה מרובע צלע א"ב כפל מרובע א"ד | |
|
וכאשר עשינו כן הוצאנו מנקדת ד' קו נכחי לקו ב"ג והוא ד"ה | |
|
ובזה נחלק המשלש לשני חלקים שוים החלק האחד משלש אד"ה והשני נוטה ד"הב"ג | |
|
דמיון שתקח מנקדת א' אשר היא ראש המשולש ה' חלקים מז' בו פחות עשירית השביעית | |
|
המשל שקו א"ב ז' אמות ומרובעו מ"ט | |
|
ונמדוד מנקדת א' ה' אמות פחות עשירית השביעית ונסמן שם נקדה | |
|
ויהיה מרובע הקו אשר הוא מנקדת א' עד הנקדה אשר סמננו כ"ד אמות וחצי והוא מחצית התשבורת של המשלש הגדול | |
|
ידענו מזה שהמשולש הקטן הוא חצי המשולש הגדול ונשאר הנוטה חציו אחר | |
| If you wish to divide it into three [equal] parts, so that they are two trapezoids and one triangle: | ואם רצית לחלקו לג' חלקים עד שיהיו שני נוטים ומשולש א' | |
|
חלק צלע הגדול על ו' ומנה מנקדת א' שהוא ראש המשולש ה' וחמישית בקרוב וסמן שם נקדה והיא ב' | |
|
והוצא מזאת הנקודה קו נכחי לתושבת המשולש הגדול והוא ב"ג | |
|
והנה יחלק אל משלש קטן ואל נוטה | |
|
ויהיה הקטן שלישית המשולש הגדול | |
|
עוד תחלק הנוטה בשני חצאים וזה כשנחלק תושבת המשולש הגדול לשני חלקים שוים ונסמן שם נקדה והיא ז' | |
|
עוד נחלק תושבת המשולש הקטן כן ונגיע קו ישר מנקדה אל נקדה | |
|
אז נחלק הנוטה לשני חלקים שוים | |
| If you wish to divide it into two equal triangles and one trapezoid: | ואם רצית לחלקו לשני משלשים שוים ולנוטה | |
|
מנה מראש המשולש אשר הוא [א' ו'][187] חלקים פחות מעט מט' בצלע וסמן שם נקדת ד' | |
|
והוצא נקדת קו נכחי מנקדת ד' אל תושבת המשולש הגדול והוא קו ד"ה | |
|
ויהיה המשלש הקטן שני חלקים מהמשולש הגדול | |
|
ונשאר נוטה ד"הב"ג החלק השלישי | |
|
ומשלש אד"ה שני חלקים | |
|
תחלק תושבת ד"ה לחצאים על נקדת ז' והוצא ממנו קו אל ראש המשולש | |
|
הנה נחלק המשלש הקטן לשנים ונמצא כל המשולש הגדול נחלק לג' [188]חלקים שוים לשני משלשי אד"ז אז"ה ולנוטה ד"הב"[ג][189] | |
| This is regarding to an equilateral triangle, or an isosceles triangle, whose different side is the base of the triangle. | וזה במשלש שוה הצלעות או שוה השוקים שתהיה צלע המתחלפ' תושבת המשולש | |
| If it is a scalene triangle, or an isosceles triangle, of which the different side is not the base of the triangle and you wish to divide it into two equal parts: | ואם היה המשולש [מתחלף][190] הצלעות או שוה השוקים ולא תהיה הצלע המתחלפת תושבת המשולש ותרצה לחלקו בשני חלקים שוים | |
|
דמיון משלש אב"ג מתחלף הצלעות או משולש ד[ה][191]"ז שוה השוקים ואין התושבת צלע המתחלפת ונרצה לחלקם בשני חלקים או בג' | |
|
תמצא תשבורת כל המשולש כמו שהודעתיך במדידת המשולשים | |
|
אח"כ תסמן בצלעו האחת נקדה ונחלקהו אל משולש נצב הזויות ואל נוטה עד שיהיה תשבורת המשולש נצב הזויות חציו או שלישיתו כפי מה שתבקש והשאר ישארו הנוטה | |
| I have already taught you the measurement of all these. | וכבר הודעתיך מדידת כל אלה | |
The Second Chapter on the Division of the quadrilateral and the Circle |
הפרק השני בחלוקת התמונות המרובעות והעגולה | |
| You already know the types of the quadrilateral shapes and how they are divided into square, rhombus, parallelogram and scalene quadrilateral; and the scalene quadrilaterals are divided into various types. | כבר ידעת מיני התמונות המרובעות ואיך יחלק אל שוה הצלעות נצב הזויות ואל שוה הצלעות ובלתי נצב הזויות ואל מרובע ארוך בלתי נצב הזויות שוה הצלעות הנכחיות ואל נוטה והנוטה מינים רבים | |
| Square | ||
| I shall start with the square, as it precedes them by nature and by degree | ואחל מהמרובע שוה הצלעות נצב הזויות כי הוא הקודם בטבע ומעלה מהם | |
|
ואומר אם תרצה לחלק מרבע אבג"ד שוה הצלעות נצב הזויות לשני חלקי' | |
|
תחלק צלע ד"ג לשני חצאים על נקדת ז' ותוציא מנקדת ז' צלע נכחי לכל אחת מצלעות א"ד ב"ג ובזה יחלק המרובע לשני חלקים שוים | |
|
והמופת על זה החלוק | |
|
כבר התבאר בתמונת א' ממאמר ו' מאקלידס שהצלעות נכחיי הצלעות כאשר היה גבהותם בשעור אחד הנה יחס קצתם אל קצתם כיחס תושבותיהם קצתם אל קצת | |
|
|
ויחס ד"ג אל ז"ג כפל א"ב | |
|
|
ד"ז מחצית ד"ג | |
|
|
גם ככה ז"ג מחצית ד"ג | |
|
ובזה המופת בעצמו יחלק גם מצלע א"ד אל ב"ג | |
|
וכן תעשה אם רצית לחלקו לג' חלקים ויותר | |
|
ואם רצית לחלקו מהזויות | |
|
תגיע זוית א' אל זוית ג' ויחלק המרבע לשנים חלקים | |
|
והמופת על החלוקה הזאת | |
|
|
כי שני משלשי אב"ג אג"ד שוים | |
|
|
כי צלע א"ב שוה לצלע ד"ג | |
|
|
וצלע ב"ג לצלע א"ד | |
|
|
וזוית אב"ג שוה לזוית אד"ג | |
|
והתושבת משותפת | |
|
אם כן שני המשולשים שוים | |
|
ואם רצית לחלקו לשלשה חלקים שוים | |
|
בדרך הראשונה כבר ידעת דרכו | |
|
אמנם בזאת הדרך השנית תעשה כך | |
|
תקח מהצלע ב' שלישיו ותגיע הזוית עד הנקודה | |
|
וככה תעשה מהצלע האחר ויחלק המרובע לג' חלקי' שוים | |
|
דמיון רצינו לחלק מרבע אבג"ד לשלשה חלקים מדרך הזויות | |
|
ויהיה כל צלע מצלעותיו י' אמות | |
|
לקחנו מצלע ד"ג ו' חלקים ושני שלישי חלק עד נקדת ה' והגענו א"ה והנה משולש [192]אד"ה נצב הזויות לשלישית המרובע | |
|
גם ככה עשינו מנקדת ב' חלקנוהו ו' חלקים ושני שלישי חלק עד נקדת ז' והגענו ג"ז והנה משולש גז"ב נצב הזויות השלישית האחד | |
|
ונשאר אהז"ג הנכחי הצלעות השלישית האחר | |
|
והמופת על זה החלוק | |
|
כבר התבאר בתמונת ל"ו ממאמר א' מאיקלידס שהשטחים הנכחיי הצלעות מהמשולשים שהם על תושבות שוות ובמה שבין שני קוים נכוחיים יהיה השטח הנכחי הצלעות כפל המשולש | |
|
והנה תושבת אזה"ג מחצית תושבת משולש גב"ז וראוי שיהיו שוים | |
|
ועוד כי שטח אזה"ג שוה לשטח בטה"ג הנכחי הצלעות ונצב הזויות כי הם תושבת אחת ובמה שבין קוים הנכוחיים | |
|
ושטח אזה"ג שלישית מרובע אבג"ד כי כן יחס תושבתו אל תושבתו | |
|
א"כ שטח אזה"ג שלישית כל המרובע | |
|
ושני המשולשים שוים | |
|
|
כי צלע א"ד כמו צלע ב"ג | |
|
|
וצלע ד"ה כמו צלע ב"ז | |
|
|
וזוית אד"ה שוה לזוית גב"ז | |
|
הנה אם כן מרובע אבג"ד נחלק לג' חלקים שוים | |
|
ואם רצית לחלקו לד' חלקים | |
|
אם מן הדרך הראשונה תוכל לחלקו בשני אופנים | |
|
האחד שתחלק צלע המרובע על ד' חלקים שוים על א"ה ועל ה"ז ועל ז"ח ועל ח"ב ותגיע ה"ט ז"כ ח"ל ויחלק המרובע לד' חלקים שוים | |
|
והמופת על החלוקה הזאת | |
|
בארתיו בחלוקתו לשני חלקים | |
|
והאופן השני שתחלקהו מהאורך לשני חלקים עוד מהרחב תחלקהו ויחלק לד' חלקים שוים | |
|
דמיון רצינו לחלק מרבע אבג"ד לד' חלקים | |
|
חלקנו צלע א"ב לשני חלקים על נקדת ה' והגענו ה"ז ויפול נכחי לא"ד ולב"ג ויחלק המרובע לב' חלקים | |
|
עוד חלקנו צלע א"ד לשנים על נקדת ח' והגענו [ח"ט] ויפול נכחי לא"ב ולד"ג ויחלק המרובע על ד' חלקים | |
|
והמופת על החלוקה הזאת | |
|
|
כי מרובע אהח"ב שוה למרובע חבד"ז | |
|
|
כי צלע א"ה שוה לצלע א"ח | |
|
כי כשתחסר מן השוה שוה יהיה הנשאר שוה | |
|
ולצלע ח"כ וכ"ה כי הם נכחיי הצלעות | |
|
וכן לג' המרובעים הנשארים | |
|
אם כן כל מרובע אבג"ד נחלק לד' מרובעים דומים למרובע אבג"ד | |
|
ואם רצית לחלקו לג' חלקים מדרך השנית כלומר מדרך הזויות | |
|
תגיע ב' אלכסוניו ויחלק לד' חלקים שוים וכל חלק יהיה משולש שוה השוקים | |
|
דמיון רצינו לחלק מרבע אבג"ד לד' חלקים שוים מדרך הזויות | |
|
הוצאנו אלכסון א"ג ואלכסון ב"ד ויחלק המרובע לד' משולשים משולש אה"ב ומשולש בה"ג ומשולש גה"ד ומשולש דה"א | |
|
|
והנה משולש אה"ב שוה למשולש דה"ג | |
|
|
כי זוית אה"ב לזוית דה"ג | |
|
|
וזוית אב"ה שוה לזוית הד"ג | |
|
|
וצלע א"ב שוה לצלע ד"ג בעצמו | |
|
|
ולזה ישוה משולש אה"ד אל משולש בה"ג | |
|
|
ומשולש אה"ד ישוה למשולש אה"ב | |
|
|
כי צלע א"ד ישוה לצלע א"ב | |
|
וצלע א"ב משותף | |
|
וזוית אה"ב שוה לזוית אה"ד כי שתיהן נצבות | |
|
ואמרי שהם שוה השוקים כי צלע המרובע הגדול מחצית הקטר וחציי הקטרים הכל שוים | |
| We have already explained the rules of division of the square [lit. the equilateral right angled quadrangle]. | [193]הנה כבר בארנו משפטי חלוקת המרובע השוה הצלעות נצב הזויות | |
| Rectangle | ||
| The same rule itself is applied to the rectangle, like this, because it is similar to it in every way and its proofs are as its proofs. | וזה המשפט בעצמו אל הנכחי הצלעות הנצב הזויות הנקרא ארוך כזה כי הוא שוה עמו בכל הדרכים ומופתיו כמופתיו | |
| Rhombus | ||
|
ואם רצית לחלק המרובע שהוא שוה הצלעות אך הוא בלתי נצב הזויות כזה לשני חלקים | |
|
מדרך הראשונה תחלק צלע א"ב לשני חלקים על נקדת ה' ותוציא מנקדת ה' קו נכחי לקו א"ד ולקו ב"ג והוא קו ה"ז ובזה נחלק המרובע לשני חלקים שוים | |
|
והמופת על החלוקה הזו | |
|
|
כי א"ה כמו ה"ב | |
|
|
וא"ד כמו ב"ג | |
|
וה"ז משותף | |
|
|
וזוית דא"ה שוה לזוית זה"ב | |
|
|
וזוית אד"ז שוה לזוית הז"ג | |
|
|
וזוית אה"ז שוה לזוית הב"ג | |
|
|
וזוית אז"ד שוה לזוית בג"ז | |
|
והשטח האחד שוה לחברו | |
|
וככה תעשה אם רצית לחלקו בג' וד' חלקים | |
|
גם אם רצית לחלקו מצלע א"ד חלקהו בכזה הדרך בעצמו ומאלה המופתים בעצמם | |
|
ואם רצית לחלקו מן דרך הזויות אם רצית לחלקו בשני חלקים שוים | |
|
תגיע אלכסון א"ג ויחלק על שני חלקים שוים | |
|
והמופת על החלוקה הזאת כי משולש אב"ג שוה למשולש אד"ג | |
|
|
צלע א"ב כמו צלע ד"ג | |
|
|
וצלע א"ד כמו צלע ב"ג | |
|
וזוית אב"ג שוה לזוית אד"ג כי הם מיתרי תושבת אחת | |
|
אם כן שני המשולשים שוים והם שני חלקי המרובע | |
|
גם ככה תעשה אם רצית לחלקו מאלכסון ב"ד בכזה המופת בעצמו | |
|
ואם רצית לחלקו לשלשה חלקים שוים אם מהדרך הראשונה | |
|
חלק צלע א"ב לג' חלקים שוים על א"ה ה"ז וז"ב והוצא קו ה"ח נכוחי לא"ד וקו ז"ט נכוחי לב"ג ויחלק המרובע לג' חלקים שוים | |
|
וכן אם רצית לחלקו לד' חלקים ויותר | |
|
והמופת על החלוקה הזאת | |
|
|
כי צלע א"ד שוה לצלע ה"ח | |
|
|
וצלע ה"ח שוה לצלע ז"ט | |
|
|
וצלע ז"ט שוה לצלע ב"ג | |
|
|
וצלע א"ה שוה לצלע ה"ז ז"ב | |
|
|
וזוית הא"ד שוה לזויות זה"ח בז"ט | |
|
|
וזוית אד"ח שוה לזוית הח"ט זט"ג | |
|
|
וזוית הח"ד שוה לזויות זט"ח בג"ט | |
|
אם כן גם השטחים נכחיי הצלעות שוים והם ג' חלקי המרובע | |
|
ואם רצית לחלקו מדרך השנית עשה כך | |
|
חלק צלע ד"ג ויהיה מנקדת ד' עד נקדת ה' שני שלישי הצלע ותגיע א"ה | |
|
עוד חלק צלע א"ב ויהיה מנקדת ב' עד נקדת ז' שני שלישי הצלע ותגיע ג"ז | |
|
ובזה נחלק המרובע לג' חלקים שוים | |
|
והמופת על החלוקה הזאת | |
|
כי יחס משולש אד"ה אל שטח אזה"ג נכחי הצלעות כחצי יחס תושבתו אל תושבתו כי הם בין שני קוים נכוחיים | |
|
וכן יחס משולש גב"ז אל שטח אזה"ג לזאת הסבה בעצמה | |
|
וחצי יחס תושבתו על תושבתו כמוהו | |
|
אם כן שני המשולשים והשטח שלשה חלקים שוים והם חלקי המרובע | |
|
ואם רצית לחלקו לד' חלקי' שוים | |
|
תחלק צלע א"ב לשני חלקים שוים על נקדת ה' | |
|
גם ככה תחלק צלע ד"ג על נקדת ז' | |
|
ותגיע א"ז וג"ה | |
|
עוד תחלק א"ה לשני חלקים שוים על נקודת ח' | |
|
גם תחלק ז"ג על נקדת ט' | |
|
ותגיע ח"ט ובזה נחלק המרובע אל ארבעה [194]חלקים שוים | |
|
והמופת על החלוקה הזאת | |
|
לפי שתושבת משולש אד"ז כתושבת שטח אהז"ג נכחי הצלעות והם בין שני קוים נכחיים יהיה השטח הזה כפל כל אחד ממשולשי אד"ז גב"ה | |
|
וכאשר חלקנו זה לשני חלקים שוים לשטח אחז"ט ולשטח חהט"ג יהיה כל אחד מהשטחים שהם חלקי השטח האחד שוה לכל אחד מהמשולשים | |
|
ושני השטחים עם שני המשולשים הם כל חלקי המרובע הנה נחלק כל המרובע לד' חלקים שוים | |
|
עוד אם רצית לחלקו לארבעה חלקים שוים [מדרך שני האלכסונים | |
|
תגיע א"ג וכן ב"ד ויפגשו על נקדת ה' ויחלק המרבע לד' חלקים שוים][195] | |
|
והמופת על החלוקה הזאת | |
|
|
כי משולש אה"ב שוה למשולש אה"ד | |
|
|
צלע א"ב שוה לצלע א"ד | |
|
וצלע א"ה משותף | |
|
|
וזוית דא"ה שוה לזוית הא"ב | |
|
לפי שצלע א"ב שוה לצלע א"ד | |
|
וצלע א"ג משותף | |
|
|
ותושבת ב"ג שוה לתושבת ד"ג | |
|
|
תהיה זוית דא"ג שוה לזוית גא"ב | |
|
וזוית גא"ד וגא"ב הן זויות דא"ה והא"ב | |
|
אם כן משולש אה"ב שוה למשולש אה"ד | |
|
|
ומשולש אה"ד שוה למשולש גה"ד | |
|
|
לפי שצלע א"ד שוה לצלע ג"ד | |
|
וצלע ד"ה משותף | |
|
|
וזוית אד"ה שוה לזוית הד"ג | |
|
לפי שצלע א"ד ממשולש אד"ב שוה לצלע ד"ג ממשולש דב"ג | |
|
וצלע ד"ב משותף | |
|
|
ותושבת א"ב שוה לתושבת ב"ג | |
|
אם כן זוית אד"ב שוה לזוית בד"ג | |
|
וזויות אד"ב ובד"ג הן זויות אד"ה והד"ג | |
|
אם כן משולש אה"ד שוה למשולש גה"ד | |
|
ובזה הדרך בעצמו יתבאר שמשולש גד"ה שוה למשולש גה"ב | |
|
אם כן המשולשים הד' שהם כל חלקי המרובע שוים | |
|
ואם רצית לחלק מדרך אחרת עשה כך | |
|
דמיון רצינו לחלק מרובע אבג"ד לד' חלקים | |
|
חלקנו צלע א"ד על נקדת ה' והיא חצי הצלע | |
|
גם חלקנו צלע ב"ג לשני חצאים על נקדת ז' | |
|
גם חלקנו צלע א"ב לשני חציים על נקדת ח' | |
|
גם חלקנו צלע ד"ג לשני חצאים על נקדת ט' | |
|
אחר כך הגענו ה"ז ח"ט | |
|
ויפגשו על נקדת כ' ויחלק המרובע לד' חלקים שוים | |
|
והמופת על החלוקה הזאת | |
|
|
לפי שצלע ה"ז נכחי לצלע ד"ג | |
|
כי [מרחקי קצוותיהם][196] שוה | |
|
|
ותושבת ג"ט שוה לתושבת ט"ד | |
|
יהיה מרובע גזכ"ט שוה למרובע טכה"ד | |
|
|
ולפי שצלע ח"ט נכחי לצלע א"ד | |
|
|
ותושבת ד"ה שוה לתושבת ה"א | |
|
יהיה מרובע טכה"ד שוה למרובע הכח"א | |
|
ובזה הדרך בעצמו ישוה להם מרובע חבז"כ | |
|
ואלה ד' מרובעי' הם כל חלקי המרובע הגדול והם שוים | |
| Parallelogram | ||
| This way of dividing the rhombus is the way of dividing the parallelogram [lit. long quadrangle that is not right-angled, but its sides are parallel] and its proofs are as its proofs. | ודרך חלוקת זה המרובע הבלתי נצב הזויות הוא דרך חלוקת המרובע הארוך הבלתי נצב הזויות הנכחי הצלעות ומופתיו כמופתיו | |
| Trapezoid | ||
| Now, we will explain the division of the trapezoids, since they are of many types: | ומעתה נבאר חלוקת הנוטים כי הם מינים הרבה | |
| Isosceles Trapezoid | ||
| We start with the trapezoid whose legs are equal, but its upper base and its bottom base are not equal, and its upper base is parallel to its bottom base, which is called truncated triangle, like this: | ונחל מן הנוטה אשר צלעיו שוים וראשו ותושבתו בלתי שוים וראשו נכח תושבתו והוא אשר יקרא משולש חתוך הראש כזה | |
| Among them there is one that is right angled, but its upper base is not parallel to its bottom base. | ויש ממנו נצב הזויות אך אין ראשו נכח תושבתו | |
|
וכאשר רצינו לחלק נוטה אבג"ד שראשו נכח תושבתו ושתי צלעותיו שוים לשני חלקים שוים | |
|
נחלק צלע א"ב לשני חלקים שוים על נקדת ה' | |
|
וכן [197]נחלק צלע ג"ד על נקדת ז' | |
|
ונגיע ה"ז ויחלק נוטה אבג"ד לשני חלקים שוים | |
|
והמופת על החלוקה הזאת | |
|
|
לפי שצלע א"ד שוה לצלע ב"ג | |
|
|
ותושבת ד"ז לתושבת ז"ג | |
|
|
וראש א"ה לראש ה"ב | |
|
וצלע ה"ז הוא משותף | |
|
אם כן נוטה אהד"ז שוה לנוטה הבז"ג והם כל נוטה אבג"ד והנה נחלק לשני חלקים שוים | |
|
ואם רצית לחלקו לג' חלקים שוים והיה הראש כמו מחצית התושבת | |
|
תחלק התושבת לשני חלקים שוים על נקדת ז' | |
|
ותגיע א"ז ב"ז ויחלק הנוטה לג' משולשים שוים | |
|
והמופת על החלוקה הזאת | |
|
ששטח א"ב ד"ז נכחי הצלעות והוא כפל משולש אד"ז כי הם על תושבת אחת ובין שני קוים נכוחיים | |
|
|
וכפל משלש אד"ז שוה לשני משולשי אד"ז אז"ב | |
|
עוד [שטח] אבג"ז שוה לכפל משולש בז"ג לסבה הזאת בעצמה | |
|
|
וכפל משולש בז"ג שוה לשני משולשי אז"ב בז"ג | |
|
א"כ גם ג' המשלשים שוים כי כשתפיל אב"ז המשותף ישאר משלש אד"ז שוה למשלש בז"ג והם כל חלקי הנוטה | |
|
אמנם אם ראש הנוטה איננו במחצית התושבת אבל עודף עליו ותרצה לחלק אותו בשלשה חלקים שוים או יהיה פוחת ממנו תעשה כך | |
|
תמצא תשברתו אחר כך חלק מספר התשבורת לג' חלקים וככה יהיה חלוקתו כלומר מספר שלישית השברים הוא שלישית הנוטה וככה כל חלק וחלק | |
|
וככה תחלק את הנוטה שהוא נצב הזויות ואין ראשו נכח תושבתו | |
| Circle | ||
|
גם אם רצית לחלק העגולה לשני חלקים שוים | |
|
תוציא אלכסון העגולה ובעברה על המרכז תחלק לשני חלקים שוים | |
|
והמופת על החלוקה הזאת כי קשת חצי העגולה שוה לקשת חציה האחר והאלכסון משותף אם כן התמונה האחת שוה לאחרת | |
|
ואם רצית לחלקה לג' חלקים שוים או ליותר כמה חלקים שתרצה | |
|
תחלק העגולה כמספר החלקי' שתרצה ותסמן נקדות כמספר החלקים על העגולה אח"כ הוצא מכל נקודה קו ישר אל המרכז והנה נחלקה העגולה על מספר החלקים שרצית | |
|
והמופת על החלוקה הזאת כי הקשתות שוות והקוים שוים כי הם יוצאים מהמקיף אל המרכז ואם כן נחלקה העגולה אל משלשים שוים אשר תושבותם קשתות שוות מהעגולה | |
The Third Section on the Measuring of Solids |
החלק השלישי במדידת הגופנים | |
The First Chapter |
הפרק הראשון | |
| Know that the solids are those that have length, width and height. | דע שהגופנים הם אשר יש להם אורך ורחב וגובה | |
|
ויש מהם אשר יקרא מחודד וכל תושבותיו משולשים כזה | |
|
והיא תמונה אשר תעלה בגובהה ותכלה אל נקדה אחת | |
|
ויש מהם אשר תושבתו מרובע וצדדיו משולשים כזה | |
|
ויפלו בגבהם אל נקדה | |
|
ויש מהם שתושבתם עולה בשטח עגול ויכלה בגבהו אל נקדה כזה | |
|
[198]ויש מהם שתושבתם רבת הזויות ותכלה אל נקדה | |
|
ויש מהם שתושבתם משולש וראשם משלש וצדדיהם המרובעים עולים על צד זוית נצבת כזה | |
|
ויש שאינם עולים על נקדה אבל נפסקים קודם שיגיעו אל הנקדה כזה | |
|
ויש מהם תמונת הכדור כזה | |
| We begin to explain the measurement of the shape, whose bottom base is quadrangular, its upper base is quadrangular, and its faces are quadrangular, rising at right angle. | ונחל לפרש מדידת התמונה שתושבתה מרובע וראש המרובע וצדדיה מרבעים עולים על זויות נצבות | |
| Cube | ||
| I say that if the length, width and the height of this shape are equal, its measurement is as follows: | ואומר שזאת התמונה אם היא שוה ארכה ורחבה וגבהה מדידתה כן | |
| We multiply its length by its width, then we multiply the product by its height and this is the volume of this shape that is called a cube. | נכפול ארכה על רחבה וההוה נכפלהו על גבהה וככה מדידת זאת התמונה והיא הנקראת מעוקב | |
|
דמיון ארכה עשרה גבהה עשרה ורחבה עשרה | |
|
כפלנו י' על י' ההוה ק' עוד כפלנו י' על ק' ההוה אלף והוא מדידת זאת התמונה | |
| Cuboid | ||
| If one of the three dimensions is greater than the others, or each is different from the other, we measure it also this way: | ואם הצד הא' מן הג' יותר מהאחרים או כל אחד לא ישוה לחברו גם כן ככה נמדדהו | |
|
דמיון האורך י' והרחב ט"ו והגובה כ' | |
|
נכפול י' על ט"ו ההוה ק"נ נכפלם על כ' ההוה ג' אלפים וזאת היא מדידתה | |
| Know that the cubit, by which we measure the solid shapes is cubit in length, cubit in width, and cubit in height, and it is called solid cubit. | ודע שהאמה אשר בה אנו מודדי' התמונות הגופניות היא אמה אורך ואמה רחב ואמה גובה וזאת תקרא אמה מוגשמת | |
| Space diagonal | ||
| The space diagonal of these shapes is drawn from one vertex at its upper base to the opposite vertex at its bottom base. | ואלכסון אלה התמונות יוצא מזויות אחת אשר בראשה אל נכח הזוית אשר בתושבתה | |
|
דמיון אם יצא בראשה מזויות מזרחי' צפונית יגיע בתושבתה אל זוית מערבית דרומית | |
| The measurement of the length of the space diagonal is as follows: | ומדידת אורך האלכסון כך | |
|
דמיון בצורת המעוקב אשר כל צלע מצלעותיו י' | |
|
נקח מרובע אלכסון התושבת ונחברהו עם מרובע הגובה ונקח שרשו והוא אורך האלכסון המבוקש | |
|
וידוע שמרובע אלכסון התושבת הוא ר' ונחברהו עם מרובע הגובה שהוא ק' עלו ש' ונקח שרשם והם י"ו ורביע וחלק א' מט"ו בקרוב | |
| We measure the space diagonal of the shapes whose length is greater than their breadth [= cuboids] also this way: by that we find the diagonal of the base, add its square to the square of the altitude, then extract the root and this is the length of the space diagonal of the shape. | גם ככה נמדוד אלכסון התמונות אשר ארכם יותר מרחבם כשנמצא אלכסון התושבת ונחבר מרובעו עם מרובע הגובה ונקח השרש והוא אורך אלכסון התמונה | |
| diagonal of the base | ||
| When we want to find the diagonal of the base, we add the square of the long side of the base to the square of its short side, then extract the root and it is the diagonal of the base. | וכאשר נרצה למצוא אלכסון התושבת נחבר מרובע צלע התושבת הארוך עם מרובע צלעו הקצר ונקח השרש והוא ארך אלכסון התושבת | |
| Prism | ||
|
ואם היתה התמונה צדדיה בלתי עולים על זויות נצבות אבל דומים למעויין או תהיינה מעויינות תמדוד תושבתה כמו שידעת במדידת הצורות האלה אחר כך מה שיצא כפלהו על הגובה והוא מדידת זאת התמונה כאלה | |
|
ואם היתה תושבתה משולש וראשה משולש וצדדיה מרובעים תמצא תשבורת המשולש אשר הוא תושבתה ותכפול אותו על הגובה והעולה היא מדידת זאת התמונה בלבד שיהיה משלש ראשה עם משולש תושבתה שוים | |
|
גם אם היתה תושבת התמונה וראשה מחומשים שוים או משושים שוים ויתר מאלה תמצא תשבורת [199]התושבת וכפלהו על הגובה והעולה היא התמונה המבוקשת | |
|
ואם היתה תושבת התמונה עגולה וראש עגולה שוה לה תמצא תשבורת העגולה ותכפלהו על הגובה והעולה הוא מדידת התמונה ההיא | |
| Pyramid | ||
| But, if the shape rises gradually and ends at one point: | אך אם התמונה עולה בהדרגה וכלה אל נקדה | |
|
אם תושבתה משולש תמצא תשבורת התושבת וכפלהו על שלישית הגובה והעולה הוא מדידת זאת התמונה | |
|
וככה אם תושבתה מרובעת או מחומשת וכדומה להם שתכפול לעולם תשבורת התושבת עם שליש הגובה או כל הגובה עם שליש תשבורת התושבת והעולה הוא המבוקש | |
| Cone | ||
|
וכן אם תושבתה עגולה תמצא תשבורת העגולה וכפלה על שלישית הגובה או על כפל אורך הגובה על שלישית התשבורת של התושבת והעולה הוא המבוקש | |
| Altitude | ||
| To know how we find the altitude of the shape, I shall tell you this matter here: | ולדעת איך נמצא גובה התמונה אודיעך ענינו פה | |
| Know that the altitude is the perpendicular that is drawn at right angles from the bottom base of the shape to its top. | דע שהגובה הוא עמוד יוצא מתושבת התמונה אל ראשה על זויות נצבות | |
|
ואם היתה תושבת התמונה עגולה | |
|
נקח חצי קטרה ונכפלה על עצמה עוד נקח גובה הצד ונכפלהו על עצמו ונוציא מרובע חצי קטרה ממרובע הצד והנשאר נקח גדרו והוא אורך הגובה | |
|
דמיון עגולה חצי קטרה ו' ומרובעו ל"ו וגובה הצד י' ומרובעו ק' | |
|
גרענו ל"ו מן ק' ונשארו ס"ד לקחנו שרשם והם ח' וככה אורך הגובה | |
|
ואם היתה התושבת של התמונה משלש שוה הצלעות | |
|
תוציא עמוד מראש משלש התושבת אל תושבתו ותחלקהו לשני חלקי' ובמקום שיתחלק שם הוא מחצית העמוד וסמן שם נקדה | |
|
עוד תחלק צלע משלש התושבת לשני חלקים שוים ובמקום שיתחלק הוא מחצית הצלע וסמן שם נקדה | |
|
אח"כ ראה מה בין נקדת מחצית העמוד לנקדת מחצית הצלע וקח מרובעו וגרעהו ממרובע גובה צד התמונה וקח שרש הנשאר והוא גובה התמונה | |
|
אך אם תושבת התמונה הוא מחומש שוה הצלעות והזויות או משושה והכלל רב הזויות וכן מרובע שוה הצלעות | |
|
והוא שתקח מחצית כל הצלעות ותקבצם יחד ותחלק על המחצית ההוא מרבע התושבת והיוצא מן החלוקה תרבעהו ותוציא את מרובעו ממרובע גובה הצד והנשאר בידך קח גדרו והוא גובה התמונה ההיא | |
| Pyramid with irregular polygonal base | ||
|
ואם לא היו הצלעות שוות כלומר צלעות התושבת גם לא הזויות אתה צריך למצוא גובה על יד כלי מעשה ואז תמצא תשבורת כל המשלשים הבלתי שוים אשר התושבת נחלקת עליהם בהוציאך קוים מזויותיה אל מרכזה ותחברם ותכפול על שלישית הגובה וההוה הוא המבוקש | |
| Truncated pyramid | ||
| However, if the shape does not end at one point, but rather ends at a triangular or square surface, or other than these shapes, provided that its upper base is smaller than the bottom base, there are other methods [for calculating its volume], which will be explained here: | אך אם התמונה אינה כלה אל נקדה אבל כלה אל שטח משלש או מרובע או זולת אלה בתמונה אשר תושבתה לבד שהראש קטן מן התושבת יש לה דרכים אחרי' יתבארו פה | |
| Such shape is called a truncated pyramid, because, if you extend its faces upwards it becomes a pyramid, and we have already explained to you the rules of the pyramid. | וזאת התמונה תקרא חתוכת הראש לפי שאם תוציא צדדיה למעלה תכלה אל מחודד וכבר בארנו לך משפטי המחודד | |
| This shape is divided into two pyramids: the large pyramid that ends at one point, and the smaller pyramid, the base of which is the upper base of the truncated pyramid, and it rises to one point. | והנה זאת התמונה תחלק אל שני מחודדים אל המחדד הגדול אשר כלה אל נקדה ואל [200]המחדד הקטן שראש התמונה חתוכת הראש תושבת לו ועולה אל הנקודה | |
|
ואם היתה תושבת בעלת ד' צלעות וככה הראש תוציא אורך הצדדים עד הנקודה למעלה ואתה יכול למדוד המחודד הגדול כמו שידעת | |
|
עוד תמדוד המחודד הקטן וגרעהו מהגדול והנשאר הוא מדידת התמונה חתוכת הראש | |
|
ולכן צריך להודיעך איך תמצא ארך הצדדים אשר הם מראש התמונה מחוץ עד הנקודה | |
|
דמיון תמונת אבג"ד חתוכת הראש ותושבתה קו א"ב והוא ו' אמות וראשה ג"ד והוא ד' אמות וגובה העמוד אשר באמצע והוא קו ה"ז י' אמות | |
|
כפול הראש לכל הגובה יעלה מ' אמות | |
|
חלק אותם על ב' אשר הם יתרון התושבת על הראש יהיו כ' והוא אורך קו ה"ט הנשאר מהגובה | |
|
רצית למצוא ארך הצדדים אשר הם למעלה מהראש עד הנקודה | |
|
תקח מרובע כ' שהם ארך ה"ט והם ת' | |
|
ומרובע ב' שהם אורך ג"ה והם ד' | |
|
וחברם עם ת' והם ת"ד גם גדרם שהם כ' אמות ועשירית אמה בקרוב והם אורך צלע ג"ט שהוא מחוץ | |
|
| ||
|
ואם רצית למצוא אורך כל צלע המחודד שהוא צלע א"ט | |
|
תקח מרובע קו ז"ט שארכו ל' ומרובעו תת"ק | |
|
גם מרובע חצי התושבת שהוא קו א"ז והוא ג' אמות ומרובעו ט' | |
|
חברם עם תת"ק יעלו תתק"ט קח גדרם והוא ל' ועשירית א' וחלק אחד מל' והוא אורך כל התשבורת של הצלע | |
|
| ||
|
תמצא תשבורת תושבת המחודד הגדול שהוא ו' על ו' אמות וההוה ל"ו | |
|
כפלם עם שלישית הגובה שהם י' כי כל הגובה הם ל' וההוה ש"ס והוא מדידת המחודד הגדול | |
|
עוד תשוב למדוד המחודד הקטן שתושבתו ד' על ד' ותשברתה הם י"ו | |
|
כפלם עם ו' וב' שלישיות שהם שליש גבהו וההוה ק"ו וב' שלישיות | |
|
תגרעם מן ש"ס שהם מדידת המחודד הגדול ישארו רנ"ג ושלישית אחד והוא מדידת התמונה חתוכת הראש המבוקשת | |
|
אך מפני שהחשבון קשה מעט נתנו בעלי החשבון דרך אחרת קצרה מזאת | |
|
והוא שתקח מרובע התושבת והוא ל"ו ומרובע הראש והוא י"ו ותכפול גדר הראש בגדר התושבת וההוה כ"ד תחבר הל"ו והי"ו והכ"ד והעולה ע"ו כפלם עם שלישית הגובה שהם ג' ושליש ההוה רכ"ג ושליש והוא מדידת התמונה חתוכת הראש | |
|
| ||
|
ואם היתה תושבת התמונה עגולה וכן ראשה | |
|
כגון תמונה אשר תושבתה עגולה וקטר העגולה ד' אמות וראש העגולה וקטרה ב' אמות | |
|
המרובע ד' אשר הוא קטר התושבת והוא י"ו ומרובע ב' שהוא קטר הראש והוא ד' וכפול ב' בד' הרי ח' והכל כ"ח | |
|
גרע [שביעיתם][201] וחלק אחד מי"ד נשארו כ"ב כפלם עם ד' שהוא שלישית הגובה יעלו פ"ח והוא מדידת התמונה הזאת | |
|
| ||
|
ולפעמים ימצא כלי אחד מחובר משתי תמונות כזאת התמונה הנזכרת שיהיו שני ראשיו עגולות קטנות ואמצעיתו רחב כזאת התמונה הנזכרת | |
|
ותמדוד כל אחת מן חלקי הכלי השנים בפני עצמו כמו שידעת וחבר שניהם וככה מדידת הכלי | |
|
ואם היה תושבת התמונה וראשה [202]משלשים שוי הצלעות אך צלעות התושבת גדולות מצלעות הראש | |
|
כגון תמונה שתושבתה משלש שוה הצלעו' כל אחת מצלעותיו [ח' אמות וראשה משלש שוה הצלעות כל אחת מצלעותיו][203] ד' אמות וגבהו ו' אמות | |
|
אתה יודע כי תשבורת משלש התושבת כ"ח פחות ב' שביעיו' האחד בקירוב וגדר המספר הזה ה' אמות וב' שביעיות | |
|
ואתה יכול לדעת הרביע מחלק רבוע המשלשים | |
|
ותמצא רבוע משלש הראש ז' אמות פחות חצי שביעית וגדר המספר הזה שנים וד' שביעיות אמה וחצי שביעית | |
|
חבר תשבורת שני המשלשים ויהיו ל"ד אמות וט' חלקים מי"ד באמה | |
|
הוסף עליהם גדר משלש התושבת בגדר משלש הראש ויהיו י"ד אמה פחות שביעית על דרך קירוב יהיה הכל מ"ח וחצי | |
|
| ||
|
מונה אותו בשליש הגובה והוא ב' יהיה הכל צ"ז והוא מדידת חתוכת הראש | |
|
| ||
| This way you know the volume of every truncated pyramid, whose bottom base is a pentagon, or other shape: | ועל הדרך הזה תדע מדידת כל תמונה חתוכת הראש אשר תושבתה מחומש או תמונה אחרת | |
| You know the areas of the two bases, add to them the product of the root of the area of the upper base by the root of the area of the bottom base, sum up all, then multiply [the sum] by a third of the altitude, and you find the volume of that shape. | והוא שתהיה יודע השני ראשים בתשברתם ותוסיף עליהם רבוע גדר תשבורת הראש בגדר תשבורת התושבת ותכלול הכל ותכפול אותו בשליש הגובה ותמצא מדידת התמונה ההיא | |
| I give you here examples of many shapes, so that you may train yourself in measuring them: | והנה אתן לך דמיון בתמונות הרבה כדי שתרגיל את עצמך במדידתם | |
| Pyramid with a square base | ||
|
תמונה מחודדת תושבתה מרובע נמדדה כך יהיה כל אחד מצלעות התושבת מכ"ד אמות וצלעות המחודד מי"ח אמות | |
|
תקח מרובע הכ"ד וההוה תקע"ו קח מחציתם והם רפ"ח | |
|
גם קח מרובע הי"ח וההוה שכ"ד | |
|
תגרע מאלה הרפ"ח נשארו ל"ו קח גדרם והם ו' וזהו שעור עמוד המחודד | |
|
ולפי שהעמוד הוא ו' נקח שלישיתו הם ב' ותכהו עם התקע"ו וההוה אלף קנ"ב וזהו תשבורת המחודד | |
|
מחודד שתושבתו מרובע אשר כל אחת מצלעות תושבתו בעל י"ב אמות וצלעות גבהותו מל"ו אמות | |
|
ונרצה למצוא העמוד | |
|
נעשה כך | |
|
נכפול צלע התושבת כלומ' הי"ב על עצמם וההוה קמ"ד | |
|
עוד נכפול הצלע האחר על עצמו וההוה קמ"ד | |
|
נחברם ביחד ההוה רפ"ח | |
|
נקח גדרם והם י"ו גם תוספת מעט וכך הוא קטר התושבת | |
|
נקח מחצית הי"ו והם ח' וחצי | |
|
ונכפלם על עצמם ההוה ע"ב ורביע | |
|
עוד נכפול הל"ו שהם צלע גבהותו על עצמם ההוה אלף רצ"ו | |
|
נגרע מאלה הע"ב ורביע וישארו אלף רכ"ד עם תוספת מעט שבם | |
|
ונקח גדרם וההוה ל"ה עם תוספת מעט שבו וכן הוא אורך העמוד | |
|
| ||
|
תכה אלה עם הקמ"ד שהם תשבורת התושבת ההוה ה' אלפים ומ' | |
|
נקח שלישית אלה ההוה אלף תר"פ וכן הוא תשבורת המוגשם | |
| The reason we take the third is that every triangular prism is divided into three pyramids, whose altitudes are equal and their bases are triangles [Elements XII.3]. | ולמה אנו לוקחים השלישית כי כל [מוגדר][204] מוגשם נחלק לג' מחודדים כשהם שוה הגובה ותושבותיהם [205]משולשים | |
| However, we based our calculation on the [quadrangular] prism and the [quadrangular] prism is divided into two triangular prisms. So, every triangular prism with the altitude of the pyramid is three times half the given pyramid that have a square base. | ואנו עשינו חשבוננו על מוגשם נכחי השטחים והמוגשם הנכחי נחלק לב' מגודרים וכל מגודר של עמוד של המחודד הוא ג' כפלי מחצית המחודד המונח והוא בעל תושבת מרובעת | |
| As Euclid has demonstrated in Book 12 [Elements XII.7]. | כן הראה זה במופת אקלידס במאמר [הי"ב][206] | |
| Truncated pyramid with a square base | ||
| A truncated square pyramid, i.e. whose top is truncated, the sides of its bottom base are ten cubits, the sides of its upper base are 2 cubits, and its lateral sides are 9 cubits; we measure it as follows: | מחודד מזונב כלומר חתוך הראש מרבע אשר צלעות תושבתו מעשר אמות וצלעות הראש מב' אמות וצלעות גבהותו מט' אמות נמדוד אותו כן | |
|
תגרע השני אמות של צלעות הראש מצלעות של התושבת וישארו ח' | |
|
תכפלם על עצמם ויהיו ס"ד | |
|
נקח מחציתם והם ל"ב | |
|
גם נכפול הט' על עצמם ויהיו פ"א | |
|
נגרעם מאלה הל"ב ישארו מ"ט | |
|
נקח גדרם והם ז' וכן הוא העמוד | |
|
| ||
|
ולפי שהעמוד ז' אמות נמצא תשבורת המוגשם כן | |
|
נחבר הב' של הראש עם הי' של התושבת ויהיו י"ב | |
|
ונקח חציים והם ו' | |
|
נכפלם על עצמם ויהיו ל"ו | |
|
|
||
|
אחר כך תגרע מהי' הב' של הראש וישארו ח' | |
|
ונקח חציים והם ד' | |
|
ונכפלם על עצמם ויהיו י"ו | |
|
ונקח שלישיתם יהיו ה' ושליש | |
|
|
||
|
הוסף אלה על הל"ו ויהיו מ"א ושליש | |
|
כפלם על הז' ויהיו רפ"ט ושליש וכן הוא התשבורת | |
|
| ||
| Pyramid with a right-angled triangular base | ||
| If you wish to measure a pyramid whose base is a right-angled triangle, it is not necessary to investigate the lateral sides in order to find the altitude, because it has a right angle. | אם תרצה למדוד מחודד אשר תושבתו משולש נצב אינו הכרח לחקור על צלעות הגבהות למצוא העמוד בהיותו בעל זוית נצבת | |
|
ויהיה אם העמוד כ"ה אמות ואם הראשונה שאצל הזוית שאצל תושבת המשולש ד' אמות ואם האחרת ה' אמות תעשה כך | |
|
כפול הד' על הה' ההוה כ' | |
|
קח חציים והם י' | |
|
כפלם על כ"ה שהוא העמוד ההוה ר"נ | |
|
קח שישיתם והם מ"א וב' שלישים | |
|
| ||
| Due to the reason that I mention, which is that every prism whose base is a triangle, which is half a square, is divided into three pyramids that have triangular bases, so is this prism. | בעבור הסבה שאזכור והיא כל מגודר אשר תושבתו משלש בחצי מרבע הוא נחלק לג' מחודדים בעלי תושבות משולשים וכן המגודר הזה | |
|
כן הראה במופת אקלידס במאמר השני | |
| The triangular prism is a half of a square [prism] and is divided into three pyramids, the result is six, [which is] a half and a third. | והמגודר הוא חצי מרובע ונחלק לג' מחודדים ההוה הוא ו' וחצי ושליש | |
| The pyramid with a triangular base is necessarily a sixth of the square [prism], so we take one sixth. | ובהכרח שהמחודד בעל תושבת משלשי שלה ששית תושבת מהמרובע ונקח הששית | |
| Pyramid with an isosceles triangular base | ||
|
ואם המשולש שוה השוקים ויהיו השוקים מי"ב אמות והתושבת ח' אמות וצלעות הגבהות מכ"ה אמות ותרצה למדוד המחודד תעשה כך | |
|
תחלק התושבת שהוא ח' אמות לחצאין ההוה ד' | |
|
כפלם על עצמם ההוה י"ו | |
|
וכפול אחת הצלעות על עצמו כלומר הי"ב על עצמם ההוה קמ"ד | |
|
גרע מאלה מרובע ד' שהם י"ו ישארו קכ"ח | |
|
קח גדרם שהם י"א ורביע וחלק מכ"ב וחלק ממ"ד וזהו השעור של העמוד אשר על תושבת המשלש שוה השוקים | |
|
| ||
|
ואם תרצה למצא התשברת תעשה כך תכפול העמוד על התושבת כלו' י"א ורביע וחלק מכ"ב וחלק ממ"ד על הח' ההוה צ' וחלק מכ"ב | |
|
כפלם על עמוד המחודד | |
|
ותמצאנו כך לפי שבכל [207]משולש אנו לוקחים חצי עמוד התושבת נקח חצי הי"א ורביע וחלק מכ"ב וחלק ממ"ד והם ה' וחצי ושמינית וחלק ממ"ד וחלק מפ"ח | |
|
נכפלם על עצמם וההוה ל"ב וחלק ממ"ד | |
|
גם נכפול צלעות הגבהות על עצמם שהם כ"ה ההווה תרכ"ה | |
|
תגרע הל"ב ורביע ההוה תקצ"ג | |
|
נקח גדרם והם כ"ד ורביע ושמיני' עם התוספת מעט וזהו שעור העמוד | |
|
| ||
|
כפול אלה על תשבורת המשולש כלו' הצ' וחלק מכ"ב ההוה אלפים וקצ"ט | |
|
נקח מאלה ששית המרובע וההוה שס"ו וחצי וזהו תשבורת המחודד | |
| Pyramid with an obtuse-angled or acute angled triangular base | ||
| If the pyramid is obtuse-angled and its base is an obtuse-angled triangle: multiply the area of the obtuse-angled triangle by the altitude, we take its third and you are left with the volume of the pyramid. | ואם המחודד יהיה נרוח הזויות ויהיה תושבת משולש נרוח הזויות תכפול תשבורת המשלש נרוח הזויות עם העמוד ונקח השליש וישאר לך תשבורת המחודד | |
| Likewise if it is acute-angled. | וכן אם יהיה חד הזויות | |
| Pyramid with a right-angled triangular base | ||
|
מחודד שתושבתו משלש נצב הזויות ויהיה עמוד ו' אמות ותושבתו ח' אמות והמיתר לזוית הנצבת י' אמות והמחודד כל אחת מצלעותיו י"ג אמות ונרצה למצוא עמודו | |
|
נעשה כך | |
|
נמצא בתחלה אלכסון העגולה המקפת המשולש שיהיה י' אמות שהוא המיתר לזוית הנצבת | |
|
ואקח חצייה והם ה' ואכפלם על עצמם וההוה כ"ה | |
|
גם אכפול הי"ג של צלע הגבהו' על עצמם וההוה קס"ט | |
|
ואגרע מאלה הכ"ה וישארו קמ"ד | |
|
ואקח גדרם והם י"ב והתשבורת נמצאה | |
|
וכן בתחלה אמצא תשבורת המשולש והוא כ"ד אמות | |
|
ואקח שלישית העמוד שהוא עמוד המחודד והם ד' | |
|
כפלם על תשבורת התושבת ויהיו צ"ו אמות וכן הוא תשבורת המחודד | |
| Pyramid with an equilateral triangular base | ||
|
מחודד העומד על משולש שוה הצלעות נמדדהו כך | |
|
יהיה כל צלע מצלעות התושבת מל' אמות וצלעות הגבהות כ' אמות | |
|
כפול הל' על עצמם וההוה תק"ת נקח שלישיתם והם ש' | |
|
גם נכפול הת' על עצמם ההוה ת' | |
|
ומאלה אגרע הש' נשארו ק' וקח גדרם והם י' וזהו אורך העמוד | |
|
| ||
|
ולפי שהעמוד י' אמות ימצא התשבורת כן | |
|
נכפול הל' על עצמם ההוה תת"ק נקח מאלה השלישית והעשירית והוא ש"צ נקח שלישית אלה והוא ק"ל | |
|
נכפול אלה על י' שהוא שעור העמוד ההוה אלף וש' וזהו תשבורת המחודד | |
|
| ||
| Pyramid with a pentagonal base | ||
|
מחודד על תושבת בעלת ה' זויות אשר כל אחת מצלעות התושבת מי"ב אמות וצלעות הגבהות מל"ה אמות ונרצה למצוא העמוד וגם תשבורת המחודד | |
|
אחוק על הבעל ה' זויות אשר הקו המקיף ס"ג אמות | |
|
ואם כן האלכסון יהיה כ' אמות | |
|
קח מאלה חציים והם י' וכפלם על עצמם עלו ק' | |
|
עוד [208]כפול הל"ה אמות של גבהות הצלעות על עצמם יעלו אלף רכ"ה | |
|
גרע מאלה הק' ישארו אלף קכ"ה קח גדרם והם ל"ג וחצי וחלק מכ"ב עם תוספת וזהו אורך העמוד | |
|
| ||
|
כפול תשבורת התושבת הבעלת ה' זויות וכן תעשה | |
|
מן הי"ב אמות של התוספת קח חציים והם ו' וכפלם על עצמם וההוה ל"ו | |
|
עוד נכפול חצי האלכסון שהם י' על עצמם וההוה ק' | |
|
גרע מאלו הל"ו ונשארו ס"ד קח גדרם והם ח' והוא שעור העמוד של המשולש | |
|
כפלם עם התושבת שהם י"ב יעלה צ"ו קח חציים והם מ"ח והם תשבורת המשלש | |
|
כפול אלה ה' פעמים אחרי שהם ה' משולשים ויהיו ר"מ אמות כפול אלה על העמוד שהוא ל"ג וחצי וחלק מכ"ב יעלו ח' אלפים ונ' | |
|
ומאלה קח ששיתם אחרי שהם ששה מגודרים ההוה אלף שמ"א וב' שלישים וזהו תשבורת המחודד | |
| We can find the diagonal also without drawing the circle, since the side of the pentagon is a square of the side of the hexagon and the side of the decagon: | ונוכל למצוא גם בלתי חקיקת העגולה את האלכסון לפי שצלע המחומש יש לו כח על צלע המשושה ועל צלע המעושר | |
|
נקח חצי הצלע כלומר חצי הי"ב ויהיו ו' נכפלם על עצמם יעלו ל"ו | |
|
גם נכפול הי"ב על עצמם יעלו קמ"ד | |
|
גרע מאלה הל"ו נשארו ק"ח קח גדרם והוא י' אמות ושליש וחלק מט"ו וזהו שעור צלע בעל שש הזויות גם זהו שעור הארך מן המרכז | |
|
| ||
| Pyramid with a hexagonal base | ||
| The measuring of a hexagonal [pyramid] is the same, without requiring the diagonal | ויהיה גם מדידת בעל השש זויות כך בלתי שאדרוש את האלכסון | |
|
כגון שיהיה מחודד בעל שש זויות ויהיה כל א' מהצלעות מי"ב אמות וצלעות הגבהות מל"ה אמות ונרצה למצוא העמוד ותשבורת המחודד נעשה כך | |
|
נכפול הי"ב על עצמם יעלו קמ"ד | |
|
והל"ה על עצמם יעלו אלף רכ"ה | |
|
גרע מאלה הקמ"ד יעלו אלף פ"א | |
|
נקח גדרם והוא ל"ב אמות וחצי ורביע ושמינית וחלק מס"ד וידענו שזהו שעור העמוד | |
|
כפול אותו על תשבורת בעל השש וקח אותו כך | |
|
לפי שבעל השש זויות יש לו ו' משולשים שוי הזויות קח תשבורת המשלש האחד וכפלהו ו' פעמים ותמצא תשבורת בעל הו' זויות | |
|
ועשה כן במשלש השוה הצלעות | |
|
תכה צלעו הראשון על עצמו יעלה קמ"ד | |
|
נקח השליש והם מ"ח גם העשירית יהיו י"ד ושליש וחלק מט"ו חברם יהיו ס"ב ושליש וחלק מט"ו | |
|
| ||
|
כפול אלה ו' פעמים אחרי שהם ו' משולשים יעלו שע"ד ורביע וחלק מט"ו | |
|
כפול אלה על העמוד שהם ל"ב וחצי ורביע ושמינית וחלק מס"ד יעלו י"ב אלף תי"ד ושליש | |
|
קח ששיתם שהוא ששית המגודר יעלו ב' אלפים ונ"ב ושליש והם שעור המחודד | |
| Pyramid with an octagonal base | ||
|
מחודד אשר תושבתו בעל ח' זויות ונרצה למדוד אותה | |
|
יהיה מחודד אשר כל צלע מצלעות תושבתו מי' אמות וצלעות גבהותו מט"ו אמות | |
|
ואם תרצה למצוא העמוד ותשבורת המחודד תעשה כך | |
|
קח חצי צלע התושבת של בעל ח' זויות כלו' מהי' חציים [209]וההוה ה' וכפלם על עצמם וההוה כ"ה כפלם בשנים וההוה נ' קח גדרם והוא ז' אמות וחלק מי"ד | |
|
הוסף על אלה חצי צלע בעל שמנה תושבות שהם ה' ויהיה הסך י"ב וחלק מי"ד כפלם על עצמם ההוה קמ"ו עם תוספת מעט | |
|
| ||
|
וכפול חצי הצלע על עצמו ההוה כ"ה חברם עם הקמ"ו וההוה קע"א גרע קי"ז וישארו נ"ד קח גדרם והם שבע ושליש וכן הוא שעור העמוד | |
|
| ||
|
והתשבורת של המחודד תמצאהו ככה | |
|
קח תשבורת תושבת בעל הח' זויות וכפלה עם העמוד ושלישית ההוה הוא אלף וקפ"א וזהו שעור אמות תשבורת מחודד בעל ח' זויות | |
| Triangular pyramid with concavities | ||
|
מחודד משולש בעל [מגרעות][210] על תושבת קשתות קטנות מחצי העגולה מן הקצה אל הקצה ומיתר הקשת שבתושבת כל אחת י' אמות והעמודים הנופלים רחב ב' אמות וצלעות הגבהות מכ' אמות | |
|
ונעשה כך | |
|
נקח חצי מיתר אחת שבתושבת ויהיו ה' נכפלם על עצמם יעלו כ"ה | |
|
והמיתר האחר שהוא י' נכפלהו על עצמו וההוה ק' | |
|
גרע מאלה הכ"ה וישארו ע"ה קח גדרם והם ח' וחצי ושמינית וחלק א' מי"ו וכך הוא השעור שמראש המשולש עד התושבת והוא העמוד | |
|
| ||
|
נקח חציים והם ד' ורביע וחלק מי"ו וחלק מל"ב כפלם על עצמם ההוה י"ו וחצי ורביעית ותשיעית עם תוספת מעט וזהו שעור חצי התושבת | |
|
| ||
|
כפול הה' על עצמם ההוה כ"ה והסך ביחד יהיו מ"ג וחצי ורביעית ותשיעית קח גדרם והוא ו' וחצי ותשיעית וכן הוא ממרכז העגולה המקפת המשלש | |
|
| ||
|
וכשתרצה למצוא העמוד תעשה כן | |
|
כפול הו' וחצי ותשיעית על עצמם ההוה מ"ג אמות וחצי ורביעית ותשיעי' | |
|
גם כפול צלעות הגבהות שהם כ' על עצמם ההוה ת' | |
|
גרע מאלה המ"ג וחצי ורביעית ותשיעית ישארו שנ"ו וחלק מי"ח קח גדרם והוא י"ח וחצי ורביעית ותשיעית וכן הוא שעור העמוד | |
|
| ||
|
כפלהו עם תושבת המשולש וכך תקחהו | |
|
הה' שהם מחצית התושבת על עמוד תושבת המשולש שהם ח' וחצי ושמינית וחלק א' מי"ו ההוה מ"ג וחצי וכן הוא שעור אמות התשבורת המשולש | |
|
כפלם על י"א וחצי ורביע ותשיעית ההוה תת"כ עם תוספת מעט וזהו תשבורת המשולש | |
|
ומזה ראוי להוציא המגרעות וכן תעשה | |
|
תחבר התושבת והעמוד הי' והב' וההוא י"ב אמות כפלם עם עמוד המחודד שהם י"א וחצי ורביע ותשיעית ההוה רכ"ו אמות כפלם על ג' לפי ששם ג' מגרעות ההוה תרע"ט | |
|
| ||
|
וכל התשלום הוא תת"כ אמות נגרע מאלה התרע"ט ונשארו קמ"א נקח מאלה הששית ההוה כ"ג ושלישים ב' אחרי שהם מהמגודר הם כ"ג וב' שלישים וזהו תשבורת התמונה | |
| It has already been explained in Book 12 of the Elements that every prism with a triangular base is divided into three equal pyramids. | וכבר התבאר במאמר הי"ב מספר היסודות שכל מגודר שתושבתו משולשת יחלק לג' מחודדים שוים | |
| It is known that every pyramid is a third of a prism that has the same sides and the same altitude. | [211]וידוע שכל מחודד הוא שלישית המגודר כשיהיו בעלי צלעות שוות וגובה שוה | |
| It is clear from this that any pyramid with any base is a third of the prism whose base is equal to its base and its altitude is equal to its altitude. | ומבואר מזה שכל מחודד שיהיה על איזו תמונה שיהיה הוא שלישית המוגשם הנכחי שתושבתו שוה לתושבתו וגבהו הוא שוה לגבהו | |
| "Mishko" | ||
| The shape called in Greek "mishko", whose upper base and bottom base are scalene, is measured as follows: | התמונה הנקראת בלשון יון מישקו והיא תמונה שהיא מתחלפת הצלעות צלעות התושבת גם הראש נמדדה כך | |
|
הגובה יהיה נ' אמות | |
|
ואם תושבת התמונה צלעה האחת הגדולה בעלת כ"ד אמות והקטן בעל י"ו אמות | |
|
ואם הראש הצלע הגדולה בעלת י"ב אמות ואם הקטנה בעלת ח' אמות | |
|
נחבר הצלעו' הגדולו' של הראש ושל התושבת כלומר הי"ב הכ"ד ההוה ל"ו | |
|
וכן חברתי הצלעות הקטנות של הראש ושל התושבת כלומר הי"ו והח' וההוה כ"ד | |
|
קח חצי הל"ו וההוה י"ח וכן חצי הכ"ד וההוה י"ב כפול אלה על י"ח וההוה רי"ו | |
|
ועוד נגרע מן הצלע הגדולה הי"ב מן הכ"ד וישארו י"ב נקח חציים והם ו' | |
|
נגרע צלע הראש מן התושבת כלומ' הצלע הקטן והם הח' מן הי"ו וישארו ח' נקח חציים ויהיו ד' | |
|
כפלם על ו' ההוה כ"ד קח השלישית והם ח' | |
|
| ||
|
ונוסיפם על הרי"ו ויהיו יחד רכ"ד | |
|
כפלם על הגובה שהם נ' ההוה י"א אלף ר' והם שעור תשבורת זאת התמונה | |
|
| ||
| We measure it this way even if it has different numbers. | וכן נמדדהו גם אם היה בעל [מספרים][212] אחרים | |
Chapter Two on the Measuring of the Spheres and Round Solids |
הפרק השני במדידת הכדורים והגופים העגולים | |
| The sphare: Euclid has defined it in Book Eleven of the Elements that "it is when the semicircle is drawn round with the diameter fixed in two points, so they do not move, and the arc, which is half the perimeter, revolves until it returns to its position; it is the circular solid. The center of the the sphare and the center of the circle are the same". | הכדור גדרו אקלידס במאמר אחד עשר מספר היסודות שהוא אשר יעבור חצי עגולה כאשר יקויים קו הקוטר בשני צירים עד שלא יסורו וסבבה הקשת אשר היא חצי הקו המקיף עד שתשוב אל מקומם והוא מוגשם העגול ומרכז הכדור ומרכז העגולה אחת | |
| Sphere | ||
| One can measure the area of the surface surrounding the sphere alone, or measure the volume of the whole solid. | והכדור יתכן למדוד התשבורת של השטח המקיף אותו לבד גם יתכן למדוד תשבורת כל גופו | |
| When we want to find the area of the surface, square the diameter of the sphere, multiply this square by three and one-seventh, and you will get the area of the surface of the sphere. | וכאשר נרצה למצוא תשבורת השטח תרבע את קטר הכדור ותכפול את המרובע הזה ג' פעמים ושביעית פעם ויעלה בידך משיחת שטח הכדור | |
| Multiply if by a sixth of the diameter and you will receive the volume of the sphere. | כפלהו בשתות הקטר ויעלה בידך תשבורת גוף הכדור | |
|
כגון ז' אמות כדור בקטרו והקו המקיף כ"ב אמות ונרצה למצוא תשבורת גופו | |
|
נעשה כך | |
|
נכפול הקטר על עצמו ויעלו מ"ט וכשתכפול זה המרובע ג' פעמים ושביעית פעם יהיה קנ"ד והן אמות שטח הכדור | |
|
כפול אותן בשתות הקטר והוא א' ושתות יהיה ק"פ פחות שלש והוא תשבורת הכדור הזה | |
|
דרך אחרת כדור אשר קטרו ז' אמות והקו המקיף כ"ב [213]אמות ונרצה למצוא תשבורת גופו | |
|
נעשה כך | |
|
נכפול הקטר על עצמו וההוה מ"ט עוד נכפול אלה על הקטר שהם ז' יעלו שמ"ג אמות נכפול אלה י"א פעם יהיו ג' אלפים תשע"ג אמות נחלקם על כ"א ויעלה החלק האחד קע"ט וב' שלישים וכן שעור תשבורת גוף הכדור | |
|
ואם תרצה למדוד השטח תעשה כך | |
|
נמצא לעולם הקטר והם במשלינו ז' ונכפלם עם המקיף שהם כ"ב ההוה קנ"ד וכן הוא תשבורת שעור שטח הכדור | |
| If you wish to calculate the air enclosed by the sphere, according to the explanation we have preceded concerning the measuring of the sphere without the thickness of the sphere walls. | אם תרצה למדוד האויר המוקף בכדור תמדוד לפי הביאור אשר הקדמנו במדידת הכדור בלתי עובי הכתלים | |
|
כגון שיהיה אורך קטר האויר מהכדור ח' אמות ועובי שתי הכתלים ב' אמות | |
|
כפול הח' אמות של קטר האויר על עצמם יעלו ס"ד כפול אלה עוד על הח' של הקטר יעלו תקי"ב אמו' כפלם על י"א יעלו ה' אלפים תרל"ב חלקם על כ"א יעלו רס"ח אמות ושביעית וחלק מן כ"א וזהו תשבורת אויר הכדור | |
| Hemisphere | ||
| If you wish to measure a hemisphere, measure it according to the explanation of the measurement of the sphere, then divide the product by 42. | אם תרצה למדוד חצי כדור תמדדהו לפי באור מדידת הכדור והנקבצים תחלקם על מ"ב | |
|
דמיון הקטר ז' אמות והקו המקיף כ"ב אמות ותרצה למצוא תשבורת גופני של חצי הכדור | |
|
תעשה כן | |
|
כפול הז' אמות של הקטר על עצמם ההוה מ"ט כפול עוד אלה על הז' אמות של הקטר ההוה שמ"ג כפול אלה על י"א וחלק העולה על מ"ב וההוה פ"ט אמות וחצי ושליש וכן הוא שעור חצי הכדור | |
| If you want to measure [the volume of] the hemisphere's airspace, measure it according to the explanation preceded regarding the measurement of the hemisphere without the thickness of the walls. | אם תרצה למדוד אויר חצי הכדור תמדדהו לפי הביאור אשר קדם במדידת תשבורת חצי הכדור בלתי עובי הכתלים | |
|
דמיון יהיה קטר אויר חצי הכדור י' אמות ועובי שתי הכתלים ד' אמות | |
|
כפל י' אמות קטר האויר לבד על עצמם וההוה ק' עוד כפול הק' על הי' הנזכרים ההוה אלף כפלם על י"א ההוה אלפים י"א חלקם על מ"ב ההוה רס"א אמות וחצי ושלישית חלק מי"ד וזהו שעור אמות אויר חצי הכדור | |
| Quarter of a sphere | ||
| If you wish to measure a quarter of a sphere, measure it according to the explanation we have preceded of the measurement of the hemisphere, then divide the product by 84. | אם תרצה למדוד רביע הכדור תמדוד לפי הבאור שהקדמנו במדידת חצי הכדור והנקבצים חלקם על פ"ד | |
|
דמיון קטר הרביע עם עובי ב' כתלים [י"ד][214] אמות | |
|
כפלם על עצמם ההוה קצ"ו כפול אלה על י"ד של הקטר הנזכר ההוה ב' אלפים תשמ"ד כפלם על י"א ההוה ל' אלף וקפ"ד חלקם על פ"ד וקח החלק האחד וההוה הוא שנ"ט ושלישית וכן הוא שעור רביע הכדור | |
| If you want to measure the airspace of the mentioned quarter and find its volume, measure it according to the explanation mentioned regarding the measurement of the quarter without the thickness of the walls. | אם תרצה למדוד אויר הרביע הנז' ולמצוא תשברתו הגופני תמדוד לפי הביאור הנזכר [215]במדידת הרביע בלתי עובי הכתלים | |
|
כלומר הי' אמות של קטר האויר ויהיו ק' עוד כפול הק' על הי' ההוה אלף כפול האלף על י"א ההוה י"א אלפים חלקם על פ"ד ההוה ק"ל אמות וחצי ושליש וחלק מי"ב וחלק מכ"ח וכן הוא שעור מדידת אויר [הרביע][216] | |
|
| ||
|
גרעם מהשנ"ט ושליש הנחברים וישארו רכ"ח אמות ורביע ושמינית וזהו תשבורת המבוקש | |
| Hemispherical pool | ||
| If you want to measure a round spherical pool that is hemispherical, I have already demonstrated to you its measurement by measuring the hemisphere. | אם רצית למדוד בריכה מעוגלת כדוריית והיא חצי כדור כבר הראיתיך מדידתו במדידת חצי הכדור | |
| Pool smaller than a hemisphere | ||
| But, if it is smaller than a hemisphere, and you know this when its depth is less than a half of its surface, measure it as follows: | אך אמנם אם היא פחותה מחצי כדור וזה תדעהו כשיהיה עמקה פחות מחצי רחב פיה תמדדה כך | |
|
כגון בריכה שרוחב פיה ו' אמות ושליש ועמקה ב' אמות | |
| First, find its diameter, as I have taught you in the fifth chapter on the measurement of the circular shapes and those that are smaller than a circle. | תמצא את קטרה בראשונה כמו שלמדת בפרק החמישי במדידת התמונות העגולות והפחותות מחצי עגולה | |
|
וכשתחשוב את קטר הבריכה הזו תמצאנו שהוא ז' אמו' | |
|
כפול הב' שהם עמק הבריכה על הז' שהם קטר הכדור יהיו י"ד כפלם ג' פעמים ושביעית פעם יהיו מ"ד והוא מדידת שטח הבריכה | |
|
כפול אותו בשתות הקטר והוא א' ושתות יעלו נ"א ושליש וזהו תשבורת גוף הבריכה | |
| Pool larger than a hemisphere | ||
| If the pool is larger than a hemisphere, you know this when its depth is greater than a half of its surface: | ואם היתה הבריכה יותר מחצי כדור וזה תדעהו כשיהיה העומק יותר ממחצית רחב פיה | |
|
כגון בריכה שרחב פיה ו' אמות ושליש ועמקה ה' אמות | |
|
כשתבקש לדעת קטר הכדור הנחצבת ממנה תמצאנו ז' | |
|
תכפול העומק שהם ה' אמות בז' אמות הקטר ההוה ל"ה כפלם ג' פעמים ושביעית פעם יהיו ק"י וזהו מדידת שטח הבריכה | |
|
כפול אותה בשתות הקטר יהיו קכ"ח ושליש וזהו תשבורת גוף הבריכה | |
| Cylinder | ||
| If you want to measure a solid called in Greek Kýlindros, which is a long rounded solid, the width of its upper base is equal to [the width of] its bottom base: | אם רצית למדוד גוף נקרא בלשון יון קלידירו והוא גוף ארוך מעוגל שוה הרחב ראשו לתושבתו | |
|
דמיון גוף כזה אשר ארכו נ' אמות והקטר ז' אמות והמקיף כ"ב אמות | |
|
ונמצא מהמקיף כמו שאנו עושים במדידת העגולה התשבורת והוא ל"ח אמות וחצי | |
|
כפול אלה על האורך שהם נ' ההוה אלף תתקכ"ה והוא תשבורת הגוף הזה | |
| Cone | ||
| If you want to measure a cone, do as follows: | אם רצית למדוד אצטוונא מחודדת תעשה כך | |
|
דמיון אצטוונא שקטר תושבתה ז' אמות והעמוד היורד מראשה ל' אמות | |
|
נמדד התושבת מהקטר הז' אמות [ונמצא תשברתה כמו שאנו עושים במדידת העגולה ויהיה התשברת ל"ח אמות וחצי | |
|
ונקח מל' אמות][217] של גובה העמוד השליש והוא י' ונכפלם על הל"ח וחצי ההוה שפ"ה אמות וזהו תשבורת הגוף של האצטוונא הנזכרת | |
|
אם רצית למצוא תשבורת אצטוונ' מחודדת [218]מקשיית ויש לו תושבת העגולה אשר הקטר מ"ב אמות וצלעות גבהות האצטוונא כל אחת[219] מע"ה אמות | |
|
ותרצה למצא העמוד תמצאהו כך | |
|
קח גובה הצלעות אשר הם ע"ה אמות וכפלם על עצמם וההווה ה' אלפים תרכ"ה | |
|
וחצי קטר התושבת אשר הם כ"א כפלם על עצמם וההווה תמ"א | |
|
גרעם מה' אלפים תרכ"ה נשארו ה' אלפים קח גדרם הם ע"ב וזהו ארך העמוד של האצטוונה | |
|
ותמצא גם תשברת העגלה והם אלף פ"ו אמות | |
|
כפלם עם שלישית העמוד שהם כ"ד ההווה כ"ו אלף ס"ד וזהו תשברת גוף האצטוונא | |
|
ואם תרצה למצא תשברת שטחה קח חצי קטר התושבת שהם כ"א וכפלם על העמוד שהם ע"ב ההווה אלף תקי"ב כפל אלה על כ"ב ההווה ל"ג אלף רס"ד קח שביעית אלה ההווה ד' אלפים תשנ"ה בקרוב וכן הוא תשברת שטח האצטוונא | |
|
| ||
| Truncated cone | ||
|
אם תרצה למדד אצטוונא מזונבת כלומר חתוכת הראש שהקטר היותר גדול שלה י' אמות והקטן ד' אמות והארך ל' אמות ותרצה למצא תשברת גופה | |
|
תעשה כך | |
|
תחבר שני הקטרים הי' והד' וההווה י"ד וקח חציים והם ז' ונחשבהו קטר | |
|
ונמצא תשברת [220]העגלה אשר קטרה ז' כמו שידעת ויהיה ל"ח אמות וחצי | |
|
תכפלם על הל' אמות של הארך וההווה אלף קנ"ה אמות והוא תשברת גוף האצטוונא | |
The Fourth Section on the Division of Solids |
החלק הרביעי בחלוקת הגופניים | |
| This section is not so necessary in world affairs and bargaining as the previous sections, but very little of it, so we will not turn to explain the division of all the shapes only those that are necessary. | זה החלק אינו כל כך צריך בענייני העולם ומקח וממכר כאשר הם החלקים העוברים כי אם מעט מזער ממנו ולכן לא נפנה לפרש חלוקת כל התמונות רק הצריכות לבד | |
| Cube | ||
|
ונאמר אם תרצה לחלק המעקב והוא אשר ארכו שוה לרחבו ולגבהו לשני חלקים | |
|
תוציא אלכסון תושבתו וחתכהו על האלכסון לשנים ויחלק לשני מגוררים | |
|
ואם רצית לחלקו לשלשה חלקים שוים | |
|
חלק צלע תושבתו לשלשה חלקים שוים על שתי נקדות וכן צלע הראש הנכחי לו וחתכהו מנקדה אל נקדה על זויות נצבות ויחלק לשלשה חלקים שוים | |
|
ואם רצית [221]לחלקו לארבעה חלקים שוים | |
|
חלקהו בתחלה לשני מגודרים עוד הוציא עמוד מזוית התושבת אל חצי מיתרה ויחלק כל אחד משני המגוררים לשנים ובזה נחלק לארבעה חלקים שוים | |
|
ובאופן אחר חלק צלע הראש לשנים וכן צלע התושבת הנכחי לו גם ככה תעשה בצלעות הגובה וחתכם על זויות נצבות ובזה נחלק המעקב לארבעה חלקים שוים | |
| Prism whose base is a rectangle | ||
| If it is a rectangle and its altitude at a right angle is different from its length and breadth, or equal to them, its division is as the division of the cube. | ואם היה מרבע ארוך נכחי הצלעות נצב הזויות וגבהו על זויות נצבות מתחלף לארכו ורחבו או שוה לו חלוקתו בחלוקת המעוקב | |
|
Prism whose base is a rhombus |
||
| If it is a rhombus, its division into two is by that you cut it at the diagonal of its bottom base that extends from vertex to vertex. | ואם היה מעויין חלוקתו לשנים הוא שתחתכנו על האלכסון של תושבתו היוצאת מן הזוית אל הזוית | |
| There is no difference whether the diagonal is drawn from an acute angle to an acute angle, or from an obtuse angle to an obtuse angle. | ואין הפרש בין אם יצא האלכסון מזוית החדה אל החדה או מן הנרחבת אל הנרחבת | |
| If you divide it into three, divide it through the sides, as you did with the cube. | ואם תחלקנו לשלשה חלקהו מהצלעות כמו שעשית במעקב | |
| If into four, divide it through the two diagonals, as you did with the cube, or by the other way mentioned there. | ואם לארבעה תחלקנו על ידי שני האלכסונים כמו שעשית במעקב או באופן האחר הנזכר שם | |
| So is the rule of division of the parallelogram. | וכן משפט חלוקת הדומה למעויין | |
| Prism whose base is triangular | ||
| If you wish to divide the prism, whose bottom base is triangular, whose upper base is triangular, and whose faces are quadrangular, into two [equal] parts: | ואם רצית לחלק המגורר שתושבתו משלשת וראשו משלש וצלעות גבהו מרבעים לשני חלקים | |
| Draw an altitude from a vertex of its bottom base to the side parallel to it that is opposite to this vertex and divide it through the altitude at right angle, so the prism is divided into two equal parts. | הוצא מן זוית תושבתו אל הצלע הנכחי לו אשר הוא מיתר לזאת הזוית עמוד וחלקהו על העמוד על זויות נצבות וכבר נחלק המגורר לשני חלקים שוים | |
| If one of the sides of the base is longer than the other sides, draw the altitude from the vertex that is opposite to that side and divide it through it. | ואם הצלע האחד מצלעות התושבת ארוך משאר הצלעות הוצא עליו העמוד מהזוית שכנגד הצלע הזה וחלקהו עליו | |
| In another way: divide the lateral sides in two and cut it through there. | ובאופן אחר חלק צלעות הגבהות לשנים וחלקהו משם | |
| If you wish to divide it into three [equal] parts, divide it into three pyramids and this is explained in Euclid's XII. | ואם רצית לחלקו בשלשה חלקים חלקהו שלשה מחדדים וזה התבאר בי"ב לאקלידס | |
| Sphere | ||
| If you want to divide the surface of the sphere do as follows: | [222]ואם רצית לחלק הכדור בשטחו תעשה כך | |
| Mark on it two opposite points that are farthest from each other of all the points that can be on the sphere and they are called the poles of the sphere. | סמן עליו שתי נקדות מקבילות היותר רחוקות זו מזו מכל הנקדות שאפשר ליפול בכדור והם יקראו קטבי הכדור | |
| Make one of the two points a center and draw a circle about half the distance between it and the other point, so they are the larger and middle circles. | ועשה נקדה אחת משתיהן מרכז וסבב עגלה כמרחק מחצית המרחק אשר בינה ובין הנקדה האחרת והיא העגלה היותר גדולה והאמצעית | |
| Divide this circle again according to the number of parts, into which you want to divide the surface of the sphere, by marking points or other signs. | עוד חלק העגלה הזאת לפי כמות החלקים שתרצה לחלוק שטח הכדור על ידי סימנים נקודות או זולתם | |
| Then, draw semicircles, each of which pass through the two poles of the sphere and through the points that are on the middle circle. | אחר כן תעגל חציי עגולות שתעברנה כל אחת על שתי קטבי הכדור ועל הנקדות שהם בעגלה האמצעי' | |
| By that the surface of the sphere is divided as you wish. | ובזה יחלק שטח הכדור לפי רצונך | |
| Do the same if you want to divide a hemisphere, or [a part of a spherical solid] greater than a hemisphere. | וככה תעשה אם תרצה לחלק חצי כדור או יותר מחציו | |
| So, the circles pass through the pole and the circumference of the semicircle that replaces the middle circle of the sphere, or the circumference of the part that is greater than a hemisphere. | ואז תעברנה העגולות על הקטב ועל שפת חצי העגלה שהוא במקום העגלה האמצעית של הכדור או על שפת החתיכה הגדולה מחצי הכדור | |
| Likewise if the part [of a spherical solid] is smaller than a hemisphere. | גם ככה אם החתיכה קטנה מחצי [223]הכדור | |
| All the divisions we have mentioned have proofs in geometry, but we did not bring them, because they are not of great need for what we intended to explain. | ובכל אלה החלוקות שהזכרנו יש מופתים בחכמת המדות ולא הבאנו אותם כי לא היה צרך גדול במה שכווננו לבאר | |
| I have taught you everything you need to know in this science. | הנה הודעתיך כל מה שצריך לדעת בזאת המלאכה | |
| To God alone be the glory and praise forever Amen. | ולאל לבדו התהלה והשבח לעולמים אמן | |
| Over and done. | תם ונשלם[224] | |
Notes
Apparatus
- ↑ 26r
- ↑ P1031 om.
- ↑ P1031 om.
- ↑ P1031 om.
- ↑ 26v
- ↑ P1031 הרשיני
- ↑ P1031 om.
- ↑ P1031 ואחרי
- ↑ P1031 om.
- ↑ 27r
- ↑ P1031 שם אשר
- ↑ P1031 om.
- ↑ P1031 להכריח
- ↑ P1031 מדרגת
- ↑ 27v
- ↑ 28r
- ↑ P1031 om.
- ↑ 28v
- ↑ P1031: הראשון
- ↑ 29r
- ↑ P1031 om.
- ↑ P1031 om.
- ↑ 29v
- ↑ P1031 העשרים
- ↑ P1031 אעשרות
- ↑ 30r
- ↑ P1031 הן
- ↑ P1031 om.
- ↑ 30v
- ↑ P1031 om.
- ↑ P1031 om.
- ↑ 31r
- ↑ P1031 om.
- ↑ P1031 om.
- ↑ 31v
- ↑ P1031 om.
- ↑ P1031 om.
- ↑ 32r
- ↑ P1031 om.
- ↑ P1031 om.
- ↑ 32v
- ↑ P1031 om.
- ↑ P1031 om.
- ↑ P1031 om.
- ↑ 33r
- ↑ P1031 om.
- ↑ P1031 om.
- ↑ P1031 om.
- ↑ P1031 om.
- ↑ 33v
- ↑ P1031 מהב'
- ↑ 34r
- ↑ 34v
- ↑ P1031: י"א
- ↑ P1031 om.
- ↑ 35r
- ↑ P1031 om.
- ↑ P1031 om.
- ↑ P1031 om.
- ↑ P1031 לקחת
- ↑ 35v
- ↑ P1031 om.
- ↑ P1031 om.
- ↑ 36r
- ↑ P1031 om.
- ↑ P1031 מרביעי
- ↑ 36v
- ↑ P1031 om.
- ↑ P1031 om.
- ↑ P1031 י"א
- ↑ P1031 marg.
- ↑ 37r
- ↑ P1031 om.
- ↑ P1031 om.
- ↑ P1031 om.
- ↑ P1031: המדה
- ↑ P1031 om.
- ↑ 37v
- ↑ P1031 om.
- ↑ P1031 om.
- ↑ P1031 om.
- ↑ 38r
- ↑ 38v
- ↑ P1031 om.
- ↑ P1031 הה'
- ↑ P1031 om.
- ↑ 39r
- ↑ P1031 ז'
- ↑ P1031 ב' שברים
- ↑ P1031 מה'
- ↑ P1031 שלישיות
- ↑ P1031 בכפל
- ↑ 39v
- ↑ P1031 om.
- ↑ P1031 om.
- ↑ P1031 המדרגות
- ↑ P1031 וי"ח
- ↑ 40r
- ↑ P1031 om.
- ↑ P1031 ורביע
- ↑ P1031 דינרים
- ↑ P1031 om.
- ↑ P1031 om.
- ↑ P1031 om.
- ↑ 40v
- ↑ P1031 om.
- ↑ P1031 marg.
- ↑ P1031 marg.
- ↑ 41r
- ↑ P1031 om.
- ↑ P1031 om.
- ↑ 41v
- ↑ P1031 marg.: אנכי צעיר יחיאל
שאלני שואל מי שיש לו סך מה והוא יודע שהרויח כל כך במאה וע"י זה נתקבץ סך המעות ההם ועתה רוצה לדעת כמה היה הקרן והריוח כל אחד לבדו
התשובה בזה שתעריך כמה הוא הריוח מהמאה ותוסיף א' ותחלק כל המעות ויצא לך הריוח והשאר הוא הקרן
המשל היה סך המעות אלף ושמנה מאות ושבעים וחמש והריוח היה כ"ה במאה
נעריך כ"ה אצל המאה והוא רביע נקח ארבעה ועוד א' שהם חמשה נחלק כל הסך לחמשה ויצא שע"ה והוא הריוח והנשאר הוא הקרן
אלא שאם יהיה הריוח כ"ג או כ"ו שאז לא יש ערך שלם כי ערך הכ"ו אצל הק' הוא ג' וכ"ב מאיים שהם י"א חמישיים נוסיף א' יהיו ד' וי"א חמישיים אז נחלק המעות בדרך חלוק השברים על דרך זה המשל היה סך המעות - ↑ 42r
- ↑ 42v
- ↑ P1031 כל ששתי
- ↑ P1031 om.
- ↑ P1031 om.
- ↑ P1031 om.
- ↑ P1031 הארץ
- ↑ P1031 המרכז
- ↑ 43r
- ↑ P1031 מהארבעה קוים
- ↑ P1031 om.
- ↑ P1031 om.
- ↑ P1031 om.
- ↑ 43v
- ↑ 44r
- ↑ 44v
- ↑ marg. וזה יתבאר לפי הנחות זה החכם מתמונת ה' ול"ד מאמר א' ולדרוש אחר שבארנו וזולת זה המקום והוא שכאשר יצא קו מזוית משלש שוה השוקים ויחלקה לשני חצאים ב"ב נז' התושבת הנה יהיה עמוד התושבת כ"א כ"ח
- ↑ 45r
- ↑ P1031: שני ש
- ↑ 45v
- ↑ 46r
- ↑ 46v
- ↑ P1031 om. marg.: שוה לב' המרבעי' ההוי' מהב' צלעו' הנשארי' הוי' מרבע הצלע האחד ממרבע' המיתר והקו
- ↑ P1031: שתי הרביעיות הג'
- ↑ 47r
- ↑ 47v
- ↑ P1031: ואשר ישאר הוא מרובע העמוד גרענו כ"ה ממרובע הצלע הדבק בו
- ↑ P1031: om.
- ↑ 48r
- ↑ P1031: האחד שבעים ואחד
- ↑ P1031 om.
- ↑ 48v
- ↑ 49r
- ↑ 49v
- ↑ om.
- ↑ P1031 א"ה
- ↑ P1031 ב"ד
- ↑ P1031 om.
- ↑ 50r
- ↑ P1031: וחמש יתחלק א'
- ↑ P1031: וחמש
- ↑ P1031 om.
- ↑ P1031 ב"ט
- ↑ P1031 om.
- ↑ P1031: ככפל השטח הנצב הזויות שהם
- ↑ P1031 om.
- ↑ P1031 om.
- ↑ P1031 om.
- ↑ P1031 om.
- ↑ 50v
- ↑ 51r
- ↑ P1031: מקצת
- ↑ P1031: ברביע
- ↑ P1031 om.
- ↑ 51v
- ↑ P1931 om.
- ↑ P1031: חצו בה
- ↑ P1031 א"ד
- ↑ P1031 om.
- ↑ 52r
- ↑ P1031: תשברתו
- ↑ 52v
- ↑ P1031 om.
- ↑ 53r
- ↑ 53v
- ↑ 54r
- ↑ P1031: וטרם שאביא המופת על כל תמונה ותמונה ועתה אחל המופת מן המחומש
- ↑ P1031: ואקים תחלה
- ↑ 54v
- ↑ 55r
- ↑ 55v
- ↑ 56r
- ↑ 56v
- ↑ P1031 om.
- ↑ 57r
- ↑ P1031 ה
- ↑ P1031 שוה
- ↑ P1031 א
- ↑ 57v
- ↑ 58r
- ↑ 58v
- ↑ P1031 om.
- ↑ P1031: מרחק תושבתו של כל א' מהם
- ↑ 59r
- ↑ 59v
- ↑ 60r
- ↑ 60v
- ↑ P1031 רביעיתם
- ↑ 61r
- ↑ P1031 om.
- ↑ P1031 om.
- ↑ 61v
- ↑ P1031 השני
- ↑ 62r
- ↑ 62v
- ↑ 63r
- ↑ P1031 מגבעות
- ↑ 63v
- ↑ P1031: משפטים
- ↑ 64r
- ↑ P1031 ד'
- ↑ 64v
- ↑ P1031 ורביע
- ↑ P1031 om.
- ↑ NY 2639 70v
- ↑ Paris end
- ↑ 71r
- ↑ 71v
- ↑ 72r
- ↑ 72v
- ↑ NY 2639: נשלם הספר חכמת המספר וחכמת המדות שחבר מרנו ורבנו החכם ב"ר מרדכי כומטיינו יצ"ו בהכ"ר אליעזר כומטיינו יעמ"ש ונשלם ע"י לי שבתי בכ"ר משה ז"ל מקנדייה שנת הרל"ח בכ"ד לחדש אדר שבח לאל יתעלה אשר לו התהלה והיכולת וזאת היא ההעתקה השנית וכתבתיהו לר' כלב המכונה אפדופילו י"ל בכ"ר אליהו יצ"ו בכ"ר יהודה
Appendix I: Glossary of Terms
| rank | מדרגה, מעלה |
Appendix II: Bibliography
Mordecai ben Eliezer Comṭino
Constantinople & Adrianople c. 1402-1482
Sefer ha-Ḥeshbon we ha-Middot
Manuscripts:
- 1) Berlin, Staatsbibliothek (Preußischer Kulturbesitz) Or. Qu. 308 (IMHM: f 1734), (15th-16th century)
- 2) London, British Library Add. 27107/6 (IMHM: f 5782), ff. 44r-80v (cat. Margo. 1016, 6); (15th-16th century) (Book 2)
- 3) New York, Jewish Theological Seminary Ms. 2616 (IMHM: f 28869), (19th century) (Book 2)
- [NY2616]
- 4) New York, Jewish Theological Seminary Ms. 2632 (IMHM: f 28885), (19th-20th century)
- [NY2632]
- 5) New York, Jewish Theological Seminary Ms. 2633 (IMHM: f 28886), (16th century)
- [NY2633]
- 6) New York, Jewish Theological Seminary Ms. 2639 (IMHM: f 28892), (1478)
- [NY2639]
- 7) Oxford, Bodleian Library MS Heb. d. 5/1 (IMHM: f 22729), ff. 1r-17r (Cat. Neub. 2774, 1); (1522)
- 8) Paris, Bibliothèque Nationale de France heb. 1031/3 (IMHM: f 15723), ff. 26r-64v (15th-16th century)
- 9) St. Petersburg, Russian National Library Evr. I 320b (IMHM: f 50984) (1495)
- 10) St. Petersburg, Russian National Library Evr. I 343-344 (IMHM: f 50961, f 41596, f 41597) (Istanbul, 1485)
The transcript of the second book is based mainly on manuscript Paris 1031
Bibliography:
- Schub, Pincus. 1932. A Mathematical Text by Mordecai Comtino, Isis vol. XVII, no. 1, (1932), pp. 54–70.
- Silberberg, Moritz. 1905–1906. Ein handschriftliches hebräisch-mathematisches Werk des Mordecai Comtino, Jahrbuch der Jüdisch-Literarischen Gesellschaft III, pp. 277–292.
- Steinschneider, Moritz. 1893–1901. Mathematik bei den Juden. Berlin-Leipzig-Frankfurt: Kaufmann, pp. 197-198 (h63-h64); repr. Hildesheim: G. Olms, 1964 and 2001.
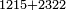
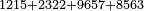
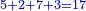
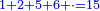
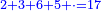

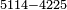
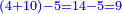
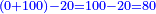
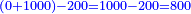
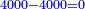
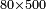




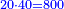
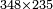
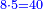
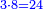
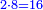
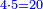


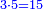







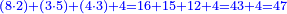
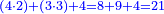

![\scriptstyle{\color{OliveGreen}{a^2=\left[\left(\frac{1}{3}\sdot a\right)^2\sdot10\right]-\left(\frac{1}{3}\sdot a\right)^2}}](/mediawiki/images/math/b/d/2/bd2b11faf7327118afeb2bc0763a2ca1.png)
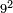
![\scriptstyle{\color{blue}{9^2=\left[\left(\frac{1}{3}\sdot9\right)^2\sdot10\right]-\left(\frac{1}{3}\sdot9\right)^2=\left(9\sdot10\right)-9=90-9=81}}](/mediawiki/images/math/d/c/6/dc6d03049d8219da7fc669593dfde1fd.png)
![\scriptstyle{\color{OliveGreen}{\left(a+1\right)^2=\left[\left(\frac{1}{3}\sdot a\right)^2\sdot10\right]-\left(\frac{1}{3}\sdot a\right)^2+a+\left(a+1\right)}}](/mediawiki/images/math/7/9/b/79b38ce6f03b950bd4495033daa3b109.png)

![\scriptstyle{\color{blue}{10^2=\left[\left(\frac{1}{3}\sdot9\right)^2\sdot10\right]-\left(\frac{1}{3}\sdot9\right)^2+\left(9+10\right)=\left(9\sdot10\right)-9+19=90-9+19=81+19=100}}](/mediawiki/images/math/0/0/5/0057acdc2e9669f94829fc55743129ac.png)
![\scriptstyle{\color{OliveGreen}{\left(a-1\right)^2=\left[\left(\frac{1}{3}\sdot a\right)^2\sdot10\right]-\left(\frac{1}{3}\sdot a\right)^2-a-\left(a+1\right)}}](/mediawiki/images/math/8/0/c/80c0ca0cf352b24ef92eec33eddcc4e1.png)
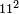
![\scriptstyle{\color{blue}{11^2=\left[\left(\frac{1}{3}\sdot12\right)^2\sdot10\right]-\left(\frac{1}{3}\sdot12\right)^2-\left(11+12\right)=\left(16\sdot10\right)-16-23=160-16-23=144-23=121}}](/mediawiki/images/math/8/5/d/85d63118acc5de20882447a88c48cec9.png)

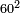
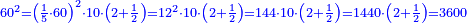
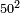
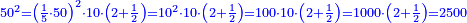
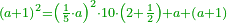
![\scriptstyle{\color{OliveGreen}{\left(a+2\right)^2=\left(\frac{1}{5}\sdot a\right)^2\sdot10\sdot\left(2+\frac{1}{2}\right)+2\sdot\left[a+\left(a+2\right)\right]}}](/mediawiki/images/math/4/8/d/48d582c5ad66bcefe735c392cb4c4701.png)
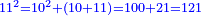
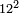
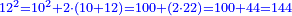

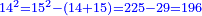
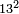

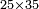
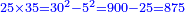

![\scriptstyle{\color{blue}{13\times14=\left[\left(3+4\right)\sdot10\right]+\left(10\sdot10\right)+\left(3\sdot4\right)=\left(7\sdot10\right)+\left(10\sdot10\right)+\left(3\sdot4\right)=70+100+12=182}}](/mediawiki/images/math/1/0/c/10c237cbc16020c219c6b4097db68c3a.png)
![\scriptstyle{\color{OliveGreen}{\sum_{k=1}^n \left[\left(\sum_{i=1}^n a_i\right)\sdot a_k\right]=\left(\sum_{i=1}^n a_i\right)^2}}](/mediawiki/images/math/9/9/f/99fd3737e42a1acf970a92fb7a977c13.png)
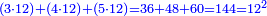
![\scriptstyle{\color{OliveGreen}{\left[\left(a+b\right)\sdot a\right]+\left[\left(a+b\right)\sdot b\right]=\left(a+b\right)^2}}](/mediawiki/images/math/8/a/a/8aae9db1c4694d4c859578e458487970.png)



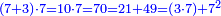
![\scriptstyle{\color{OliveGreen}{\left(a+b\right)^2=a^2+b^2+\left[2\sdot\left(a\sdot b\right)\right]}}](/mediawiki/images/math/d/f/2/df26d1cdaf50844081193e48666b1eeb.png)
![\scriptstyle{\color{blue}{3^2+7^2+\left[2\sdot\left(3\sdot7\right)\right]=9+49+\left(2\sdot21\right)=9+49+42=100=10^2=\left(3+7\right)^2}}](/mediawiki/images/math/a/a/7/aa76f2ff07aa1dec6d3c46d015817f63.png)
![\scriptstyle{\color{OliveGreen}{\left(a\sdot b\right)+\left[\left[\frac{1}{2}\sdot\left(a+b\right)\right]-a\right]^2=\left(a\sdot b\right)+\left[b-\left[\frac{1}{2}\sdot\left(a+b\right)\right]\right]^2=\left[\frac{1}{2}\sdot\left(a+b\right)\right]^2}}](/mediawiki/images/math/7/0/c/70cf1effca1e2303b584ecf2bb737846.png)
![\scriptstyle{\color{blue}{\scriptstyle\left(3\sdot7\right)+\left[\left(\frac{1}{2}\sdot10\right)-3\right]^2=\left(3\sdot7\right)+\left(5-3\right)^2}}](/mediawiki/images/math/0/3/d/03dfdce3ec288d58b8f094e10b1397f2.png)
![\scriptstyle{\color{blue}{\left(3\sdot7\right)+\left[7-\left(\frac{1}{2}\sdot10\right)\right]^2=\left(3\sdot7\right)+\left(7-5\right)^2=\left(3\sdot7\right)+2^2=21+4=25=5^2=\left(\frac{1}{2}\sdot10\right)^2}}](/mediawiki/images/math/0/6/b/06bc86c67148dd7b41b0715d8e16b65a.png)
![\scriptstyle{\color{OliveGreen}{\left[\left(a+b\right)\sdot b\right]+\left(\frac{1}{2}\sdot a\right)^2=\left[\left(\frac{1}{2}\sdot a\right)+b\right]^2}}](/mediawiki/images/math/a/9/2/a92046fe5abf282c91d09104586c53a7.png)
![\scriptstyle{\color{blue}{\left[2\sdot\left(10+2\right)\right]+\left(\frac{1}{2}\sdot10\right)^2=\left(2\sdot12\right)+5^2=24+25=49=7^2=\left(5+2\right)^2=\left[\left(\frac{1}{2}\sdot10\right)+2\right]^2}}](/mediawiki/images/math/8/1/8/818e21e090d00b6643f2d405697773bf.png)
![\scriptstyle{\color{OliveGreen}{\left(a+b\right)^2+a^2=2\sdot\left[\left(a+b\right)\sdot a\right]+b^2}}](/mediawiki/images/math/0/3/a/03a8d96192812ea7db90d5166ad5177a.png)
![\scriptstyle{\color{blue}{10^2+7^2=100+49=149=140+9=\left[2\sdot\left(7\sdot10\right)\right]+3^2}}](/mediawiki/images/math/7/3/0/730c333f2c3cd5673b8c62296b570f67.png)
![\scriptstyle{\color{OliveGreen}{4\sdot\left[\left(a+b\right)\sdot a\right]+b^2=\left[\left(a+b\right)+a\right]^2}}](/mediawiki/images/math/c/a/f/caf2f89aa2a00ea5e45e6eed3d4bc694.png)
![\scriptstyle{\color{blue}{\scriptstyle\left[4\sdot\left(10\sdot7\right)\right]+3^2=280+9=289=17^2=\left(10+7\right)^2}}](/mediawiki/images/math/2/d/9/2d930b3cf54ef8cfc52718dc74fc4c81.png)
![\scriptstyle{\color{OliveGreen}{a^2+b^2=2\sdot\left[\left[\frac{1}{2}\sdot\left(a+b\right)\right]^2+\left[a-\left[\frac{1}{2}\sdot\left(a+b\right)\right]\right]^2\right]}}](/mediawiki/images/math/3/7/c/37cd2d64c6505f872e3604129685d3bc.png)
![\scriptstyle{\color{blue}{7^2+3^2=58=2\sdot\left(25+4\right)=2\sdot\left(5^2+2^2\right)=2\sdot\left[5^2+\left(7-5\right)^2\right]=2\sdot\left[\left(\frac{1}{2}\sdot10\right)^2+\left[7-\left(\frac{1}{2}\sdot10\right)\right]^2\right]}}](/mediawiki/images/math/9/9/a/99a17ded1fe72199555d6cbb2166b760.png)
![\scriptstyle{\color{OliveGreen}{\left(a+b\right)^2+b^2=2\sdot\left[\left(\frac{1}{2}\sdot a\right)^2+\left[\left(\frac{1}{2}\sdot a\right)+b\right]^2\right]}}](/mediawiki/images/math/0/1/f/01f5c12dc0db0f20f362991f00bed1d8.png)
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle\left(10+2\right)^2+2^2&\scriptstyle=12^2+2^2=144+4=148=2\sdot74=2\sdot\left(25+49\right)=2\sdot\left(5^2+7^2\right)=2\sdot\left[5^2+\left(5+2\right)^2\right]\\&\scriptstyle=2\sdot\left[\left(\frac{1}{2}\sdot10\right)+\left[\left(\frac{1}{2}\sdot10\right)+2\right]^2\right]\\\end{align}}}](/mediawiki/images/math/e/b/8/eb8f69d48765173e3615403e795a39d7.png)

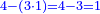
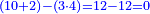
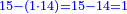
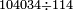
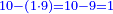
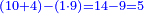
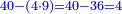
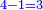
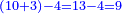
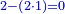
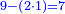

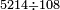
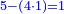



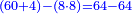
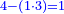
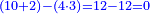
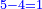
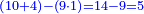
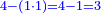
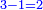
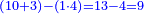
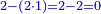
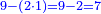
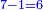
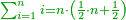
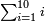
![\scriptstyle{\color{blue}{\sum_{i=1}^{10} i=\left[10\sdot\left(\frac{1}{2}\sdot10\right)\right]+\left(\frac{1}{2}\sdot10\right)=\left(10\sdot5\right)+5=50+5=55}}](/mediawiki/images/math/9/c/0/9c0ccf419889ae2f9ab62ec4b8e4cdcf.png)
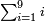
![\scriptstyle{\color{blue}{\sum_{i=1}^{9} i=9\sdot\left[\left(\frac{1}{2}\sdot9\right)+\frac{1}{2}\right]=9\sdot\left[\left(4+\frac{1}{2}\right)+\frac{1}{2}\right]=9\sdot5=45}}](/mediawiki/images/math/3/9/8/398b018782593241c093b22cca24e4c5.png)
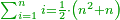
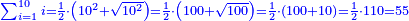
![\scriptstyle{\color{OliveGreen}{\sum_{i=1}^{n} i=\frac{1}{2}\sdot\left[n\sdot\left(n+1\right)\right]}}](/mediawiki/images/math/7/b/1/7b1fe2c816cc633f5b118cec023fbb52.png)
![\scriptstyle{\color{blue}{\sum_{i=1}^{10} i=\frac{1}{2}\sdot\left[10\sdot\left(10+1\right)\right]=\frac{1}{2}\sdot\left(10\sdot11\right)=\frac{1}{2}\sdot110=55}}](/mediawiki/images/math/f/0/b/f0beea7728e8dfdf06ae991f6f9f4969.png)
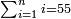

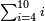

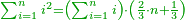
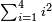
![\scriptstyle{\color{blue}{\sum_{i=1}^{4} i^2=\left(\sum_{i=1}^{4} i\right)\sdot\left(\frac{2}{3}\sdot4+\frac{1}{3}\right)=\left[4\sdot\left(\frac{1}{2}\sdot4+\frac{1}{2}\right)\right]\sdot3=10\sdot3=30}}](/mediawiki/images/math/d/6/4/d64cc07c12c0138acae5e059090777d3.png)

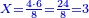
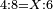

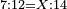
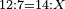
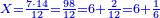
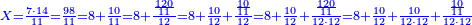
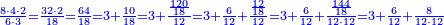
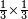
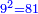
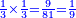

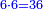

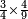
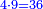
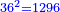

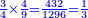
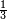



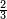
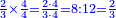
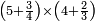

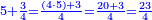
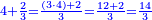
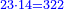

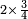
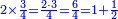
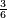

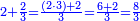
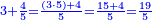
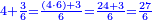

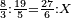



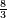
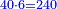

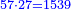
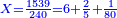


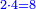
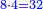
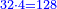
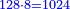

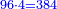
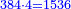
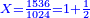
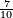

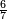
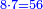
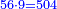

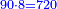



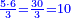
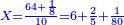
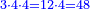
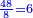
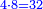

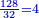
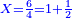
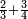
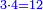
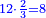
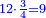
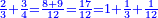
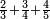
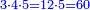
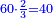
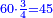

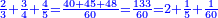
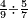
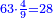

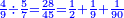
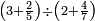
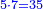
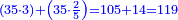
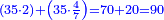
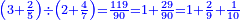
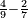
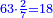
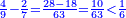
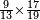
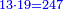

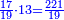
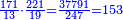
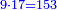
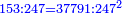

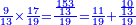
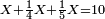


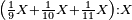

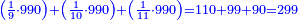
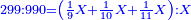
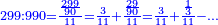

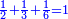
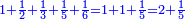
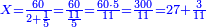
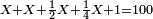
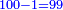
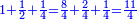

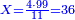
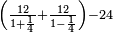
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle\left(\frac{12}{1+\frac{1}{4}}+\frac{12}{1-\frac{1}{4}}\right)-24&\scriptstyle=\left(\frac{4\sdot12}{5}+\frac{4\sdot12}{3}\right)-24=\left(\frac{48}{5}+\frac{48}{3}\right)-24=\left[\left(9+\frac{1}{2}+\frac{1}{10}\right)+16\right]-24\\&\scriptstyle=\left(25+\frac{1}{2}+\frac{1}{10}\right)-24=1+\frac{1}{2}+\frac{1}{10}\\\end{align}}}](/mediawiki/images/math/1/e/7/1e7936713ac4ef992265d97656b4527d.png)
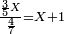
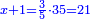
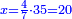
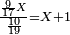
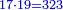
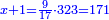
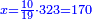
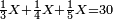

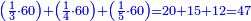

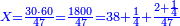

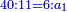
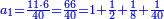

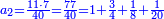
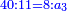
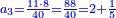
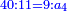


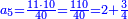
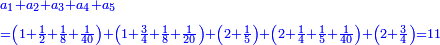
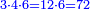
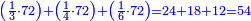
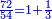
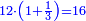

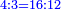



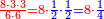
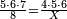
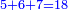
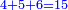

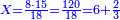
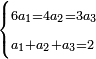

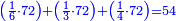
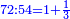
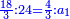

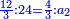
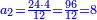
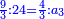
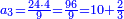
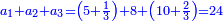
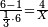
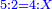
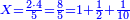
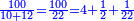 hours
hours
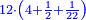
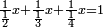
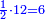 ] that are 8 measures [
] that are 8 measures [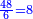 ] in one hour.
] in one hour.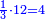 ] that are 12 measures [
] that are 12 measures [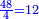 ] in one hour.
] in one hour. ] that are 16 measures [
] that are 16 measures [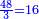 ] in one hour.
] in one hour.
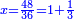

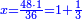
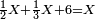


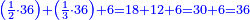
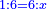

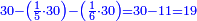
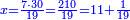

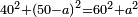

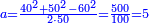

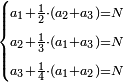
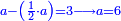
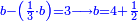
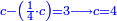
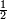
![\scriptstyle{\color{blue}{\left(2\sdot6\right)+\left[2\sdot\left(4+\frac{1}{2}\right)\right]+\left(2\sdot4\right)=12+9+8=29}}](/mediawiki/images/math/4/b/6/4b603e8d3bbbfa2a95c54b43550e6b90.png)
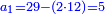
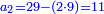
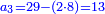
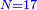
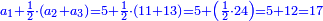
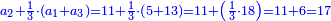
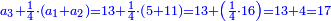
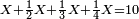
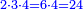
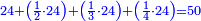



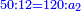
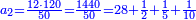
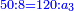
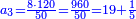

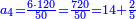
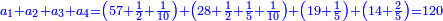

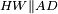 ;
; 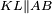
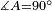
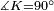
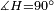
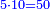

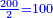


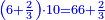
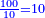
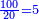
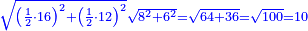
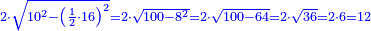
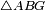 is equilateral and the height that is drawn from the top of the triangle, which is point A, to its base falls on point D. I say that point D is the midpoint of the base.
is equilateral and the height that is drawn from the top of the triangle, which is point A, to its base falls on point D. I say that point D is the midpoint of the base.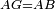
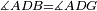 , because both are right angles.
, because both are right angles.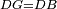
 are the whole base, so each of them is half the base.
are the whole base, so each of them is half the base.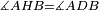 , because both are right angles.
, because both are right angles.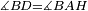
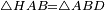
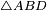 is half the rectangle HADB and it is half
is half the rectangle HADB and it is half 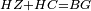


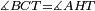
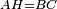 because both are half the height.
because both are half the height.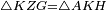
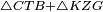 , which is the whole rectangle, is equal to trapezoid TKBG +
, which is the whole rectangle, is equal to trapezoid TKBG + 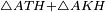 , which is the whole
, which is the whole ![\scriptstyle{\color{blue}{\frac{\frac{1}{2}\sdot\left[\left(18^2+16^2\right)-20^2\right]}{16}=\frac{\frac{1}{2}\sdot\left[\left(324+256\right)-400\right]}{16}=\frac{\frac{1}{2}\sdot\left(580-400\right)}{16}=\frac{\frac{1}{2}\sdot180}{16}=\frac{90}{16}=5+\frac{1}{2}+\frac{1}{8}}}](/mediawiki/images/math/f/7/3/f73435d35ad5400be494c8303131c9e3.png)
![\scriptstyle{\color{blue}{\frac{\frac{1}{2}\sdot\left[\left(20^2+16^2\right)-18^2\right]}{16}=\frac{\frac{1}{2}\sdot\left[\left(400+256\right)-324\right]}{16}=\frac{\frac{1}{2}\sdot\left(656-324\right)}{16}=\frac{\frac{1}{2}\sdot332}{16}=\frac{166}{16}=10+\frac{1}{3}+\frac{1}{24}}}](/mediawiki/images/math/d/e/e/dee0caefa78f17b2cef3bcf2cd2a4f40.png)
![\scriptstyle{\color{blue}{\frac{\frac{1}{2}\sdot\left[\left(13^2+14^2\right)-15^2\right]}{14}=\frac{\frac{1}{2}\sdot\left(365-225\right)}{14}=\frac{\frac{1}{2}\sdot140}{14}=\frac{70}{14}=5}}](/mediawiki/images/math/7/8/3/783a551c01b9ea613ff5c0eabfac1652.png)
![\scriptstyle{\color{blue}{\frac{\frac{1}{2}\sdot\left[\left(15^2+14^2\right)-13^2\right]}{14}=\frac{\frac{1}{2}\sdot\left(421-169\right)}{14}=\frac{\frac{1}{2}\sdot252}{14}=\frac{126}{14}=9}}](/mediawiki/images/math/b/f/7/bf766cb8e6b3c557c5d462a068fd9fb8.png)
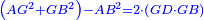
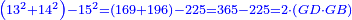
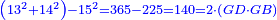
![\scriptstyle{\color{blue}{\frac{1}{2}\sdot\left[\left(AG^2+GB^2\right)-AB^2\right]=GD\sdot GB}}](/mediawiki/images/math/7/4/8/748508ebd173b2cc55dfe28afe598058.png)
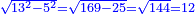
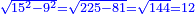
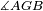 is the obtuse angle. I say that the square of AB, which is the side opposite to the obtuse angle, exceeds [the sum of] the square of AG and GB by twice the product of GB by GD.
is the obtuse angle. I say that the square of AB, which is the side opposite to the obtuse angle, exceeds [the sum of] the square of AG and GB by twice the product of GB by GD.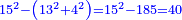
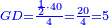
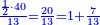
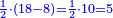
![\scriptstyle{\color{blue}{\left[\frac{1}{2}\sdot\left(8+18\right)\right]\sdot12=\left(\frac{1}{2}\sdot26\right)\sdot12=13\sdot12=156}}](/mediawiki/images/math/e/a/0/ea08993541dcdb6a714ee276acb87ff0.png)
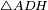
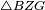 is the product of the height by half ZG.
is the product of the height by half ZG.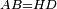
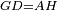

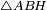 is isosceles and each of its sides is known, height AZ is also known and it is 12 cubits, as previously known.
is isosceles and each of its sides is known, height AZ is also known and it is 12 cubits, as previously known.

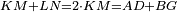
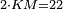
![\scriptstyle{\color{blue}{\left[\frac{1}{2}\sdot\left(27+6\right)\right]\sdot12=\left(16+\frac{1}{2}\right)\sdot12=198}}](/mediawiki/images/math/9/d/e/9de6313c2fd3f200764fd0870f5a2185.png)
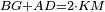
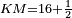 cubits.
cubits.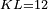 cubits, because so is line AZ.
cubits, because so is line AZ.![\scriptstyle{\color{blue}{\left[\frac{1}{2}\sdot\left(17+6\right)\right]\sdot12=\left(\frac{1}{2}\sdot23\right)\sdot12=\left(11+\frac{1}{2}\right)\sdot12=138}}](/mediawiki/images/math/7/4/8/748dbe89048135b7801384e661ea0d80.png)
 is obtuse, line AH is 12 cubits.
is obtuse, line AH is 12 cubits.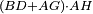 is double the trapezoid ABGD.
is double the trapezoid ABGD.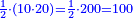
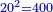
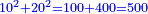
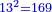

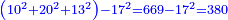
![\scriptstyle{\color{blue}{\frac{1}{2}\sdot\left[\left(10^2+20^2+13^2\right)-17^2\right]=\frac{1}{2}\sdot380=190}}](/mediawiki/images/math/4/3/b/43beba494c89b2c59109ae39f2e9c4df.png)
![\scriptstyle{\color{blue}{\left[\frac{1}{2}\sdot\left[\left(10^2+20^2+13^2\right)-17^2\right]\right]^2=190^2=36100}}](/mediawiki/images/math/d/3/2/d32b40bae389ab2b11098e28e104034e.png)
![\scriptstyle{\color{blue}{\frac{\left[\frac{1}{2}\sdot\left[\left(10^2+20^2+13^2\right)-17^2\right]\right]^2}{10^2+20^2}=\frac{36100}{500}=72+\frac{1}{5}}}](/mediawiki/images/math/7/e/7/7e79a9d986890aa1aeed4f4f699036a9.png)
![\scriptstyle{\color{blue}{13^2-\frac{\left[\frac{1}{2}\sdot\left[\left(10^2+20^2+13^2\right)-17^2\right]\right]^2}{10^2+20^2}=169-\left(72+\frac{1}{5}\right)=96+\frac{1}{2}+\frac{1}{5}+\frac{1}{10}}}](/mediawiki/images/math/2/f/f/2ff4a286617161ba6538fe16cd9340f1.png)
![\scriptstyle{\color{blue}{\left[13^2-\frac{\left[\frac{1}{2}\sdot\left[\left(10^2+20^2+13^2\right)-17^2\right]\right]^2}{10^2+20^2}\right]\sdot\left(10^2+20^2\right)=\left(96+\frac{1}{2}+\frac{1}{5}+\frac{1}{10}\right)\sdot500=48400}}](/mediawiki/images/math/7/e/9/7e9c6104a753be345410bd51bea66300.png)
![\scriptstyle{\color{blue}{\frac{1}{2}\sdot\sqrt{\left[13^2-\frac{\left[\frac{1}{2}\sdot\left[\left(10^2+20^2+13^2\right)-17^2\right]\right]^2}{10^2+20^2}\right]\sdot\left(10^2+20^2\right)}=\frac{1}{2}\sdot\sqrt{48400}=\frac{1}{2}\sdot220=110}}](/mediawiki/images/math/3/e/0/3e012163af7ea7eb69192f5c8acba60d.png)
 is 100 cubits.
is 100 cubits.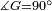 , then
, then 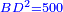
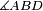 is acute angle.
is acute angle.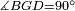
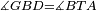
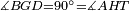
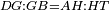
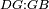 is known, therefore
is known, therefore 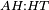 is also known.
is also known.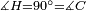
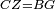 , therefore the whole AZ is known.
, therefore the whole AZ is known.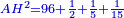
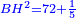
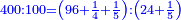
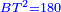
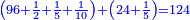

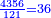
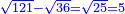

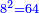
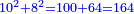
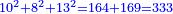
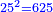
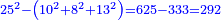
![\scriptstyle{\color{blue}{\frac{1}{2}\sdot\left[25^2-\left(10^2+8^2+13^2\right)\right]=\frac{1}{2}\sdot292=146}}](/mediawiki/images/math/e/c/c/ecc7586dd5f035eea6f671d3d5081e76.png)
![\scriptstyle{\color{blue}{\left[\frac{1}{2}\sdot\left[25^2-\left(10^2+8^2+13^2\right)\right]\right]^2=146^2=21316}}](/mediawiki/images/math/a/5/7/a577a8cc66f154ab7d983ad2e8945cd1.png)
![\scriptstyle{\color{blue}{\frac{\left[\frac{1}{2}\sdot\left[25^2-\left(10^2+8^2+13^2\right)\right]\right]^2}{10^2+8^2}=\frac{21316}{164}=129+\frac{160}{164}}}](/mediawiki/images/math/a/c/e/ace687e014ef4c62a1d24f2f3cdd18b7.png)
![\scriptstyle{\color{blue}{13^2-\frac{\left[\frac{1}{2}\sdot\left[25^2-\left(10^2+8^2+13^2\right)\right]\right]^2}{10^2+8^2}=169-\left(129+\frac{160}{164}\right)=39+\frac{4}{164}}}](/mediawiki/images/math/2/6/8/2684f199f49f71fe83bbd250bb2984a0.png)
![\scriptstyle{\color{blue}{\begin{align}&\scriptstyle\frac{1}{2}\sdot\sqrt{\left[13^2-\frac{\left[\frac{1}{2}\sdot\left[25^2-\left(10^2+8^2+13^2\right)\right]\right]^2}{10^2+8^2}\right]\sdot\left(10^2+8^2\right)}\\&\scriptstyle=\frac{1}{2}\sdot\sqrt{\left(39+\frac{4}{164}\right)\sdot164}=\frac{1}{2}\sdot\sqrt{6400}=\frac{1}{2}\sdot80=40\\\end{align}}}](/mediawiki/images/math/4/6/c/46c10d9784ea8604b1e595cd2f1bcd0e.png)
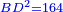

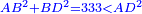
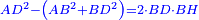

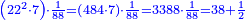
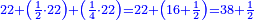
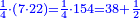
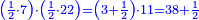


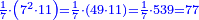
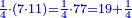
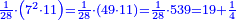
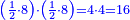
![\scriptstyle{\color{blue}{\left[\frac{1}{2}\sdot\left(12+4\right)\right]\sdot4=\left(\frac{1}{2}\sdot16\right)\sdot4=8\sdot4=32}}](/mediawiki/images/math/8/d/8/8d83c1ccc4fe29eae359fd6c484142c0.png)
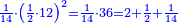
![\scriptstyle{\color{blue}{\left[\left[\frac{1}{2}\sdot\left(12+4\right)\right]\sdot4\right]+\left[\frac{1}{14}\sdot\left(\frac{1}{2}\sdot12\right)^2\right]=32+\left(2+\frac{1}{2}+\frac{1}{14}\right)=34+\frac{1}{2}+\frac{1}{14}}}](/mediawiki/images/math/2/2/e/22e6843edb5629234c27d85e0f6a6b4d.png)
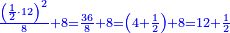
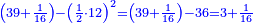
 .
.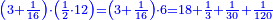
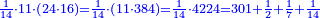
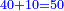
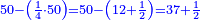
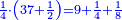
![\scriptstyle{\color{blue}{\left[\left(40+10\right)-\frac{1}{4}\sdot\left(40+10\right)\right]+\frac{1}{4}\sdot\left[\left(40+10\right)-\frac{1}{4}\sdot\left(40+10\right)\right]=\left(37+\frac{1}{2}\right)+\left(9+\frac{1}{4}+\frac{1}{8}\right)=46+\frac{3}{4}+\frac{1}{8}}}](/mediawiki/images/math/5/4/6/546f676ef4b9d67272730622885886d3.png)

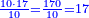
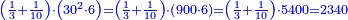
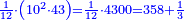
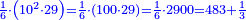


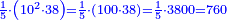
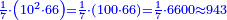
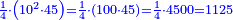
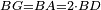 , then
, then 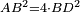
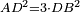
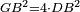

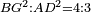
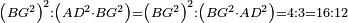

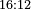
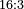
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle\sqrt{\left(\frac{1}{3}+\frac{1}{24}\right)\sdot\left[\frac{1}{2}\sdot\left(10^2\right)^2\right]}&\scriptstyle=\sqrt{\left(\frac{1}{3}+\frac{1}{24}\right)\sdot\left(\frac{1}{2}\sdot100^2\right)}\\&\scriptstyle=\sqrt{\left(\frac{1}{3}+\frac{1}{24}\right)\sdot\left(\frac{1}{2}\sdot10000\right)}=\sqrt{1875}\approx43+\frac{1}{3}+\frac{1}{38}+\frac{1}{40}+\frac{1}{41}\\\end{align}}}](/mediawiki/images/math/a/8/b/a8b3e8303b37799967d7f710d961660a.png)

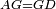 .
.
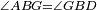
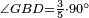
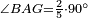
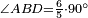
 is an angle of a pentagon.
is an angle of a pentagon.

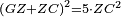
 .
.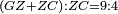
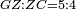
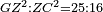

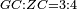
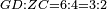
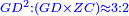
 , which is a fifth of pentagon ABGDH.
, which is a fifth of pentagon ABGDH.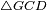 is equilateral and its side is known.
is equilateral and its side is known.![\scriptstyle{\color{blue}{\sqrt{\left[\frac{1}{4}\sdot\left(10^2\right)^2\right]\sdot27}=\sqrt{\left(\frac{1}{4}\sdot100^2\right)\sdot27}=\sqrt{\left(\frac{1}{4}\sdot10000\right)\sdot27}=\sqrt{2500\sdot27}=\sqrt{67500}\approx259}}](/mediawiki/images/math/4/d/3/4d3bdac682ce6ffdd58bcb8ea53b6bd7.png)
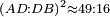
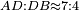
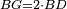
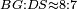
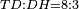
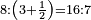
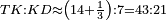
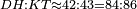
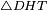 is
is  .
.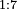
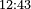
 equal to it.
equal to it.
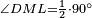

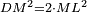
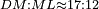

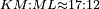
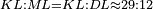
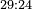 .
. .
. is
is 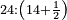 .
.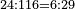
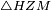 of the nonagon is known.
of the nonagon is known.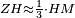
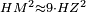

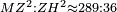

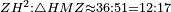
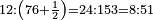

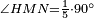
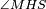 equal to it.
equal to it.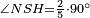
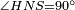
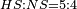
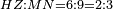
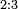 .
.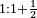 .
.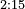
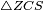 is ²/₁₁ of the hendecagon.
is ²/₁₁ of the hendecagon.
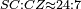
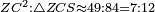
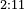 , the ratio of the square on ZC to the hendecagon is
, the ratio of the square on ZC to the hendecagon is 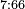
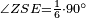
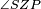 equal to it.
equal to it.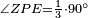

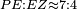
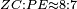
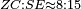
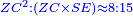
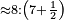
 , as they are two corresponding angles between parallel lines, when a straight line falls on them, and the exterior angle is equal to its corresponding angle.
, as they are two corresponding angles between parallel lines, when a straight line falls on them, and the exterior angle is equal to its corresponding angle. , for the same reason itself.
, for the same reason itself.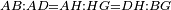
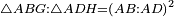
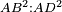 .
.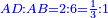
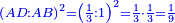
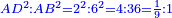
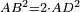

![\scriptstyle{\color{blue}{\left[5-\left(\frac{1}{10}\sdot\frac{1}{7}\right)\right]^2\approx24+\frac{1}{2}}}](/mediawiki/images/math/e/6/f/e6f954ad0509a9e00070461f3136ffc7.png)
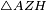 and the trapezoid DHBG.
and the trapezoid DHBG.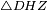 an isosceles triangle, but the base is not the different side, and we want to divide them into two or three parts:
an isosceles triangle, but the base is not the different side, and we want to divide them into two or three parts: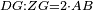
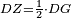
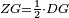

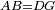
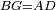
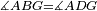
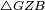 is one-third [of the square].
is one-third [of the square]. , but they should be equal.
, but they should be equal.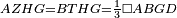
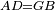

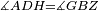
 ,
,  ,
,  ,
,  .
.


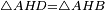
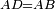
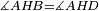 as both are right angles.
as both are right angles.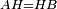
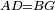
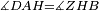
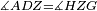
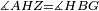


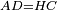
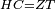
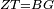
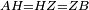
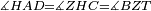
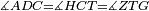
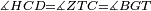
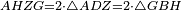
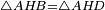
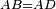
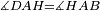
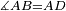
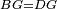
 and
and  are
are  and
and 
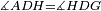
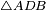 is equal to DG of
is equal to DG of 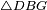
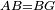
 and
and  are
are  and
and 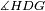




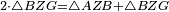
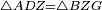 remains and they are all the parts of the trapezoid.
remains and they are all the parts of the trapezoid.

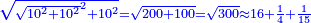

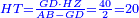

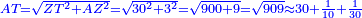
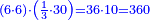
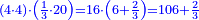
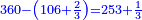
![\scriptstyle{\color{blue}{\left[6^2+4^2+\left(6\sdot4\right)\right]\sdot\left(\frac{1}{3}\sdot10\right)=\left(36+16+24\right)\sdot\left(3+\frac{1}{3}\right)=76\sdot\left(3+\frac{1}{3}\right)=253+\frac{1}{3}}}](/mediawiki/images/math/c/e/6/ce63e386ef65503bd3c5888990558e47.png)
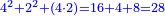
![\scriptstyle{\color{blue}{\left[\left[4^2+2^2+\left(4\sdot2\right)\right]-\left[\left[4^2+2^2+\left(4\sdot2\right)\right]\sdot\left(\frac{1}{7}+\frac{1}{14}\right)\right]\right]\sdot\left(\frac{1}{3}\sdot12\right)=\left[28-\left[28\sdot\left(\frac{1}{7}+\frac{1}{14}\right)\right]\right]\sdot4=22\sdot4=88}}](/mediawiki/images/math/2/b/6/2b604c49ea587ec240c8db56055c8195.png)

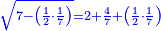
![\scriptstyle{\color{blue}{\left(28-\frac{2}{7}\right)+\left[7-\left(\frac{1}{2}\sdot\frac{1}{7}\right)\right]+\left[\sqrt{28-\frac{2}{7}}\sdot\sqrt{7-\left(\frac{1}{2}\sdot\frac{1}{7}\right)}\right]=\left(28-\frac{2}{7}\right)+\left[7-\left(\frac{1}{2}\sdot\frac{1}{7}\right)\right]+\left(14-\frac{1}{7}\right)=48+\frac{1}{2}}}](/mediawiki/images/math/1/5/7/157501bac8e8587cbb10d16187fde90c.png)
![\scriptstyle{\color{blue}{\left[\left(28-\frac{2}{7}\right)+\left[7-\left(\frac{1}{2}\sdot\frac{1}{7}\right)\right]+\left[\sqrt{28-\frac{2}{7}}\sdot\sqrt{7-\left(\frac{1}{2}\sdot\frac{1}{7}\right)}\right]\right]\sdot\left(\frac{1}{3}\sdot6\right)=\left(48+\frac{1}{2}\right)\sdot2=97}}](/mediawiki/images/math/d/b/1/db12d412ae287a496fec4608880231a5.png)
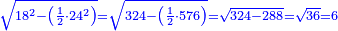

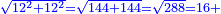
![\scriptstyle{\color{blue}{\sqrt{36^2-\left[\frac{1}{2}\sdot\left(16+\right)\right]^2}=\sqrt{36^2-\left(8+\frac{1}{2}\right)^2}=\sqrt{1296-\left(72+\frac{1}{4}\right)}=\sqrt{1224+}=35+}}](/mediawiki/images/math/a/d/9/ad976f00757975e4d9e26432b8e1ea14.png)
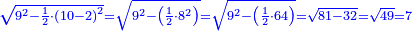
![\scriptstyle{\color{blue}{\left[\frac{1}{2}\sdot\left(2+10\right)\right]^2=\left(\frac{1}{2}\sdot12\right)^2=6^2=36}}](/mediawiki/images/math/b/2/4/b2458bff7c27d0dc621756937c322dc0.png)
![\scriptstyle{\color{blue}{\frac{1}{3}\sdot\left[\frac{1}{2}\sdot\left(10-2\right)\right]^2=\frac{1}{3}\sdot\left(\frac{1}{2}\sdot8\right)^2=\left(\frac{1}{3}\sdot16\right)=5+\frac{1}{3}}}](/mediawiki/images/math/7/f/6/7f6d4ba7462cf8de2a87c8880b5298a4.png)
![\scriptstyle{\color{blue}{\left[\left[\frac{1}{2}\sdot\left(2+10\right)\right]^2+\frac{1}{3}\sdot\left[\frac{1}{2}\sdot\left(10-2\right)\right]^2\right]\sdot7=\left[36+\left(5+\frac{1}{3}\right)\right]\sdot7=\left(41+\frac{1}{3}\right)\sdot7=289+\frac{1}{3}}}](/mediawiki/images/math/d/3/d/d3d9a6d51896998616e58e3ca9e8393d.png)
![\scriptstyle{\color{blue}{\frac{1}{6}\sdot\left[\left[\frac{1}{2}\sdot\left(4\sdot5\right)\right]\sdot25\right]=\frac{1}{6}\sdot\left[\left(\frac{1}{2}\sdot20\right)\sdot25\right]=\frac{1}{6}\sdot\left(10\sdot25\right)=\frac{1}{6}\sdot250=41+\frac{2}{3}}}](/mediawiki/images/math/1/3/c/13ce602347a2c0b86a591749a333d20b.png)
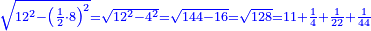
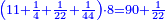
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle\sqrt{25^2-\left[\frac{1}{2}\sdot\left(11+\frac{1}{4}+\frac{1}{22}+\frac{1}{44}\right)\right]^2}&\scriptstyle=\sqrt{25^2-\left(5+\frac{1}{2}+\frac{1}{8}+\frac{1}{44}+\frac{1}{88}\right)^2}=\sqrt{625-\left(32+\frac{1}{44}\right)}\\&\scriptstyle=\sqrt{593}=24+\frac{1}{4}+\frac{1}{8}+\end{align}}}](/mediawiki/images/math/1/8/6/18653ed83078f9459e14ef5c51954d35.png)
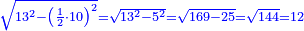
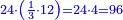
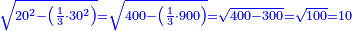
![\scriptstyle{\color{blue}{\left[\frac{1}{3}\sdot\left[\left(\frac{1}{3}+\frac{1}{10}\right)\sdot30^2\right]\right]\sdot10=\left[\frac{1}{3}\sdot\left[\left(\frac{1}{3}+\frac{1}{10}\right)\sdot900\right]\right]\sdot10=\left(\frac{1}{3}\sdot390\right)\sdot10=130\sdot10=1300}}](/mediawiki/images/math/1/0/f/10ff2874527bf72809b882277f0e1e56.png)
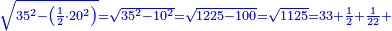
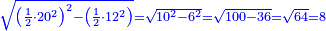

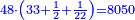
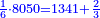
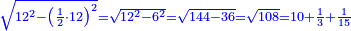
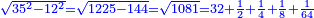
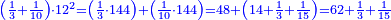
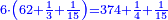
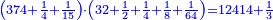
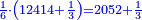
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle\left[\sqrt{2\sdot\left(\frac{1}{2}\sdot10\right)^2}+\left(\frac{1}{2}\sdot10\right)\right]^2&\scriptstyle=\left(\sqrt{2\sdot5^2}+5\right)^2=\left(\sqrt{2\sdot25}+5\right)^2=\left(\sqrt{50}+5\right)^2\\&\scriptstyle=\left[\left(7+\frac{1}{14}\right)+5\right]^2=\left(12+\frac{1}{14}\right)^2=146+\end{align}}}](/mediawiki/images/math/7/0/8/70856a90276917f921a2e6a8bdd63be7.png)
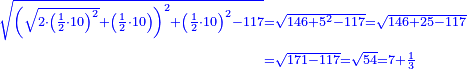

![\scriptstyle{\color{blue}{\left[\frac{1}{2}\sdot\left(8+\frac{1}{2}+\frac{1}{8}+\frac{1}{16}\right)\right]^2=\left(4+\frac{1}{4}+\frac{1}{16}+\frac{1}{32}\right)^2=16+\frac{1}{2}+\frac{1}{4}+\frac{1}{9}+}}](/mediawiki/images/math/7/7/9/779acdb7cd08e06a81b97e4a8c56ab36.png)
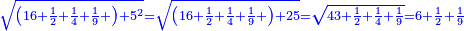
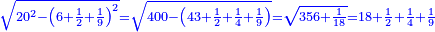
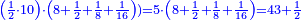

![\scriptstyle{\color{blue}{3\sdot\left[\left(10+2\right)\sdot\left(18+\frac{1}{2}+\frac{1}{4}+\frac{1}{9}\right)\right]=3\sdot\left[12\sdot\left(18+\frac{1}{2}+\frac{1}{4}+\frac{1}{9}\right)\right]=3\sdot226=679}}](/mediawiki/images/math/d/b/7/db7d38b302d88c4d4817baa1dbaeaa7b.png)

![\scriptstyle{\color{blue}{\left[\frac{1}{2}\sdot\left(12+24\right)\right]\sdot\left[\frac{1}{2}\sdot\left(16+8\right)\right]=\left(\frac{1}{2}\sdot36\right)\sdot\left(\frac{1}{2}\sdot24\right)=\left(18\sdot12\right)=216}}](/mediawiki/images/math/f/a/4/fa44a56090e19950596fc53b53bcf9eb.png)
![\scriptstyle{\color{blue}{\frac{1}{3}\sdot\left[\left[\frac{1}{2}\sdot\left(24-12\right)\right]\sdot\left[\frac{1}{2}\sdot\left(16-8\right)\right]\right]=\frac{1}{3}\sdot\left[\left(\frac{1}{2}\sdot12\right)\sdot\left(\frac{1}{2}\sdot8\right)\right]=\frac{1}{3}\sdot\left(6\sdot4\right)=\left(\frac{1}{3}\sdot24\right)=8}}](/mediawiki/images/math/f/6/b/f6b2f5acadf9bb789f4bec03ee5a20ee.png)
![\scriptstyle{\color{blue}{\begin{align}&\scriptstyle\left[\left[\left[\frac{1}{2}\sdot\left(12+24\right)\right]\sdot\left[\frac{1}{2}\sdot\left(16+8\right)\right]\right]+\frac{1}{3}\sdot\left[\left[\frac{1}{2}\sdot\left(24-12\right)\right]\sdot\left[\frac{1}{2}\sdot\left(16-8\right)\right]\right]\right]\sdot50\\&\scriptstyle=\left(216+8\right)\sdot50=224\sdot50=11200\\\end{align}}}](/mediawiki/images/math/e/c/5/ec5e79deb67c642a168c44cb46b3845d.png)
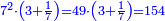
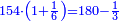
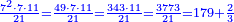
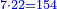
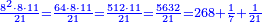
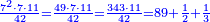


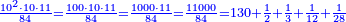
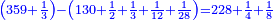
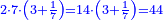
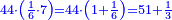
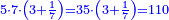


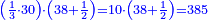
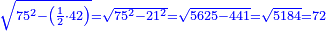

![\scriptstyle{\color{blue}{\frac{1}{7}\sdot\left[\left(\frac{1}{2}\sdot42\right)\sdot72\sdot22\right]=\frac{1}{7}\sdot\left(21\sdot72\sdot22\right)=\frac{1}{7}\sdot\left(1512\sdot22\right)=\frac{1}{7}\sdot33264\approx4755}}](/mediawiki/images/math/3/c/0/3c06fa38cad1b6ded072dacc71951d98.png)