Difference between revisions of "ספר הכללים במספר"
(→Geometrical Problems) |
(→Additional Problems - MS Mantova) |
||
| (446 intermediate revisions by the same user not shown) | |||
| Line 1: | Line 1: | ||
| − | + | {{#annotpage: time="1300-1400", peshat_title="00002044"}} | |
| + | __TOC__ | ||
<div class="center" style="width: auto; margin-left: auto; margin-right: auto;"><big>'''ספר הכללים במספר'''</big></div> | <div class="center" style="width: auto; margin-left: auto; margin-right: auto;"><big>'''ספר הכללים במספר'''</big></div> | ||
{| | {| | ||
|- | |- | ||
| | | | ||
| − | == Problems of Various Types == | + | == <span style=color:green>Problems of Various Types</span> == |
| | | | ||
|- | |- | ||
| − | |<span style=color: | + | |<span style=color:green>[Gold Weights: 1 kikkar = 100 liṭra; 1 liṭra = 20 dinar/zuz; 1 dinar/zuz = 12 pešiṭim/pešuṭim]</span> |
| + | | | ||
| + | |- | ||
| + | | | ||
| + | === <span style=color:green>Find a Number Problem - Subtraction of Fractions</span> === | ||
| + | |||
| | | | ||
|- | |- | ||
| − | + | ! | |
| − | <math>\scriptstyle\frac{1}{a}-\frac{1}{b}=\frac{\left[\frac{1}{a}\sdot\left(a\sdot b\right)\right]-\left[\frac{1}{b}\sdot\left(a\sdot b\right)\right]}{a\sdot b}</math> | + | <math>\scriptstyle{\color{OliveGreen}{\frac{1}{a}-\frac{1}{b}=\frac{\left[\frac{1}{a}\sdot\left(a\sdot b\right)\right]-\left[\frac{1}{b}\sdot\left(a\sdot b\right)\right]}{a\sdot b}}}</math> |
| | | | ||
|- | |- | ||
| | | | ||
| − | :{{#annot:⅓-¼|678|Sdu0}}1) | + | :{{#annot:⅓-¼|678|Sdu0}}1) If a man asks you: how large is the third from the quarter? |
:<math>\scriptstyle\frac{1}{3}-\frac{1}{4}</math> | :<math>\scriptstyle\frac{1}{3}-\frac{1}{4}</math> | ||
| − | |style="text-align:right;"|א | + | |style="text-align:right;"|‫<ref>88r</ref>א <big>אם</big> ישאלך אדם כמה הוא יותר השליש מן הרביע{{#annotend:Sdu0}} |
|- | |- | ||
| | | | ||
| − | :<span style=color: | + | :<span style=color:green>'''common denominator:'''</span> say: 3 times 4 is 12. |
| − | |style="text-align:right;"|אמור ג' פעמים ד הם י"ב | + | :<math>\scriptstyle{\color{blue}{3\sdot4=12}}</math> |
| + | |style="width:45%; text-align:right;"|אמור ג' פעמים ד' הם י"ב | ||
|- | |- | ||
| | | | ||
| + | :The third is 4 dinar. | ||
| + | |style="text-align:right;"|השליש הוא ד' דנריצימו | ||
| + | |- | ||
| + | | | ||
| + | :The quarter is 3 dinar. | ||
| + | |style="text-align:right;"|הרביע הוא ג' דנריציסמו | ||
| + | |- | ||
| + | | | ||
| + | :We find that the third is greater than the quarter by a twelfth. | ||
:<math>\scriptstyle{\color{blue}{\frac{1}{3}-\frac{1}{4}=\frac{\left(\frac{1}{3}\sdot12\right)-\left(\frac{1}{4}\sdot12\right)}{12}=\frac{4-3}{12}=\frac{1}{12}}}</math> | :<math>\scriptstyle{\color{blue}{\frac{1}{3}-\frac{1}{4}=\frac{\left(\frac{1}{3}\sdot12\right)-\left(\frac{1}{4}\sdot12\right)}{12}=\frac{4-3}{12}=\frac{1}{12}}}</math> | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|נמצא שהשליש הוא יותר מן הרביע א' תריציסמו |
| − | |||
| − | נמצא שהשליש הוא יותר מן הרביע א' תריציסמו | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:¼-⅕|678|hEgl}} | + | *{{#annot:¼-⅕|678|hEgl}}If you are asked: how large is the quarter from the fifth? |
:<math>\scriptstyle\frac{1}{4}-\frac{1}{5}</math> | :<math>\scriptstyle\frac{1}{4}-\frac{1}{5}</math> | ||
|style="text-align:right;"|וכן אם ישאלך כמה יותר הרביע מן החומש{{#annotend:hEgl}} | |style="text-align:right;"|וכן אם ישאלך כמה יותר הרביע מן החומש{{#annotend:hEgl}} | ||
|- | |- | ||
| | | | ||
| − | :<span style=color: | + | :<span style=color:green>'''common denominator:'''</span> do as we did above and say: 4 times 5 is 20. |
| + | :<math>\scriptstyle{\color{blue}{4\sdot5=20}}</math> | ||
|style="text-align:right;"|תעשה כמו שעשינו למעלה ואמור ד' פעמים ה' הם כ‫' | |style="text-align:right;"|תעשה כמו שעשינו למעלה ואמור ד' פעמים ה' הם כ‫' | ||
|- | |- | ||
| | | | ||
| + | :The quarter is 5-twentieths. | ||
| + | |style="text-align:right;"|הרביע הוא ה' בינטיני | ||
| + | |- | ||
| + | | | ||
| + | :The fifth is 4-twentieths. | ||
| + | |style="text-align:right;"|החומש הוא ד' בינטיני | ||
| + | |- | ||
| + | | | ||
| + | :We find that the quarter is greater than the fifth by a twentieth. | ||
:<math>\scriptstyle{\color{blue}{\frac{1}{4}-\frac{1}{5}=\frac{\left(\frac{1}{4}\sdot20\right)-\left(\frac{1}{5}\sdot20\right)}{20}=\frac{5-4}{20}=\frac{1}{20}}}</math> | :<math>\scriptstyle{\color{blue}{\frac{1}{4}-\frac{1}{5}=\frac{\left(\frac{1}{4}\sdot20\right)-\left(\frac{1}{5}\sdot20\right)}{20}=\frac{5-4}{20}=\frac{1}{20}}}</math> | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|נמצא שהרביע הוא יותר מן החומש א' בינטינו |
| − | + | |- | |
| − | נמצא שהרביע הוא יותר מן החומש א' בינטינו | + | |Likewise for everything similar to it. |
| − | וכן כל הדומה לו | + | |style="text-align:right;"|וכן כל הדומה לו |
|- | |- | ||
| | | | ||
| − | *{{#annot:⅓-⅕|678|t2Xm}} | + | *{{#annot:⅓-⅕|678|t2Xm}}If you are asked: how large is the third from the fifth? |
:<math>\scriptstyle\frac{1}{3}-\frac{1}{5}</math> | :<math>\scriptstyle\frac{1}{3}-\frac{1}{5}</math> | ||
|style="text-align:right;"|וכן אם ישאלך כמה הוא יותר השליש מן החמישית{{#annotend:t2Xm}} | |style="text-align:right;"|וכן אם ישאלך כמה הוא יותר השליש מן החמישית{{#annotend:t2Xm}} | ||
|- | |- | ||
| | | | ||
| − | :<span style=color: | + | :<span style=color:green>'''common denominator:'''</span> say: 3 times 5 is 15. |
| + | :<math>\scriptstyle{\color{blue}{3\sdot5=15}}</math> | ||
|style="text-align:right;"|אמור ג' פעמים ה' הם ט"ו | |style="text-align:right;"|אמור ג' פעמים ה' הם ט"ו | ||
|- | |- | ||
| | | | ||
| + | :The third is 5-fifteenths. | ||
| + | |style="text-align:right;"|השליש הוא ה' קוויניציסמי | ||
| + | |- | ||
| + | | | ||
| + | :The fifth is 3-fifteenths. | ||
| + | |style="text-align:right;"|והחומש הוא ג' קוויניציס | ||
| + | |- | ||
| + | | | ||
| + | :We find that the third is greater than the fifth by two-fifteenths. | ||
:<math>\scriptstyle{\color{blue}{\frac{1}{3}-\frac{1}{5}=\frac{\left(\frac{1}{3}\sdot15\right)-\left(\frac{1}{5}\sdot15\right)}{15}=\frac{5-3}{15}=\frac{2}{15}}}</math> | :<math>\scriptstyle{\color{blue}{\frac{1}{3}-\frac{1}{5}=\frac{\left(\frac{1}{3}\sdot15\right)-\left(\frac{1}{5}\sdot15\right)}{15}=\frac{5-3}{15}=\frac{2}{15}}}</math> | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|נמצא שהשליש הוא יותר מן החומש ב' קוויניציסמו |
| − | |||
| − | נמצא שהשליש הוא יותר מן החומש ב | ||
|- | |- | ||
| − | | | + | | |
| + | |||
| + | === <span style=color:green>Find a Number Problem - Addition of Fractions</span> === | ||
| + | |||
| | | | ||
|- | |- | ||
| Line 67: | Line 102: | ||
:{{#annot:⅓+¼+⅕+⅙+⅐|677|753y}}2) How much are a third, a quarter, a fifth, a sixth, and a seventh of one pašuṭ? | :{{#annot:⅓+¼+⅕+⅙+⅐|677|753y}}2) How much are a third, a quarter, a fifth, a sixth, and a seventh of one pašuṭ? | ||
:<math>\scriptstyle\frac{1}{3}+\frac{1}{4}+\frac{1}{5}+\frac{1}{6}+\frac{1}{7}</math> | :<math>\scriptstyle\frac{1}{3}+\frac{1}{4}+\frac{1}{5}+\frac{1}{6}+\frac{1}{7}</math> | ||
| − | |style="text-align:right;"|ב | + | |style="text-align:right;"|ב <big>אם</big> ישאלך אדם כמה הוא שליש פשוט ורביע וחומש ושתות ושביעית{{#annotend:753y}} |
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''common denominator:'''</span> you should divide the pašuṭ into the number in which you find all these fractions. | |
|style="text-align:right;"|תצטרך לחלק הפשוט לכל כך חלקי' שתמצא בו כל אלו השעורים | |style="text-align:right;"|תצטרך לחלק הפשוט לכל כך חלקי' שתמצא בו כל אלו השעורים | ||
|- | |- | ||
| | | | ||
| − | :: | + | :Follow this way and say: 3 times 4 is 12; 5 times 12 is 60; 6 times 60 is 360; 7 times 360 is 2520; in this number you find your question. |
|style="text-align:right;"|וכלך לדרך זו ואמור ג' פעמים ד' הם י"ב<br> | |style="text-align:right;"|וכלך לדרך זו ואמור ג' פעמים ד' הם י"ב<br> | ||
ה' פעמין י"ב הם ס‫'<br> | ה' פעמין י"ב הם ס‫'<br> | ||
ו' פעמי' ס' הם ש"ס<br> | ו' פעמי' ס' הם ש"ס<br> | ||
ז' פעמין ש"ס הם אלפי' ותק"כ ובזה השעור תמצא שאלתך | ז' פעמין ש"ס הם אלפי' ותק"כ ובזה השעור תמצא שאלתך | ||
| + | |- | ||
| + | |colspan="2"| | ||
| + | :<math>\scriptstyle{\color{blue}{\left[\left[\left(3\sdot4\right)\sdot5\right]\sdot6\right]\sdot7=\left[\left(12\sdot5\right)\sdot6\right]\sdot7=\left(60\sdot6\right)\sdot7=360\sdot7=2520}}</math> | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\frac{1}{3}=\frac{\frac{1}{3}\sdot2520}{2520}=\frac{840}{2520}}}</math> | + | :The third is 849 parts. |
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{3}=\frac{\frac{1}{3}\sdot2520}{2520}=\frac{840}{2520}}}</math> | ||
|style="text-align:right;"|השליש הוא תת"מ חלקי‫' | |style="text-align:right;"|השליש הוא תת"מ חלקי‫' | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\frac{1}{4}=\frac{\frac{1}{4}\sdot2520}{2520}=\frac{630}{2520}}}</math> | + | :The quarter is 630 parts. |
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{4}=\frac{\frac{1}{4}\sdot2520}{2520}=\frac{630}{2520}}}</math> | ||
|style="text-align:right;"|הרביע הוא תר"ל חלקי‫' | |style="text-align:right;"|הרביע הוא תר"ל חלקי‫' | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\frac{1}{5}=\frac{\frac{1}{5}\sdot2520}{2520}=\frac{504}{2520}}}</math> | + | :The fifth is 504 parts. |
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{5}=\frac{\frac{1}{5}\sdot2520}{2520}=\frac{504}{2520}}}</math> | ||
|style="text-align:right;"|החומש הוא תק"ד חלקי‫' | |style="text-align:right;"|החומש הוא תק"ד חלקי‫' | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\frac{1}{6}=\frac{\frac{1}{6}\sdot2520}{2520}=\frac{420}{2520}}}</math> | + | :The sixth is 420 parts. |
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{6}=\frac{\frac{1}{6}\sdot2520}{2520}=\frac{420}{2520}}}</math> | ||
|style="text-align:right;"|השתות הוא ת"כ חלקי‫' | |style="text-align:right;"|השתות הוא ת"כ חלקי‫' | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\frac{1}{7}=\frac{\frac{1}{7}\sdot2520}{2520}=\frac{360}{2520}}}</math> | + | :The seventh is 360 parts. |
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{7}=\frac{\frac{1}{7}\sdot2520}{2520}=\frac{360}{2520}}}</math> | ||
|style="text-align:right;"|השבעית הוא ש"ס חלקי‫' | |style="text-align:right;"|השבעית הוא ש"ס חלקי‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | :The total is 2754. |
| − | |style="text-align:right;"|הרי שמן כולם הם אלפי' ותשנ"ד חלקי‫' | + | |style="text-align:right;"|הרי שמן כולם הם אלפי' ותשנ"ד חלקי‫' |
| − | תוציא מהם אלפים ותק"כ חלקי' שהוא אחד שלם נשארו בידך רל"ד חלקי‫' | + | |- |
| − | + | | | |
| − | + | :Subtract 2520 parts from it, which is one unit; you are left with 234 parts. | |
| + | |style="text-align:right;"|תוציא מהם אלפים ותק"כ חלקי' שהוא אחד שלם נשארו בידך רל"ד חלקי‫' | ||
|- | |- | ||
| − | |||
| | | | ||
| + | :We find that a third, a quarter, a fifth, a sixth, and a seventh of one pašuṭ are one pašuṭ and 234 parts of one pašuṭ, when you divide it into 2520 parts, which is 117 parts of 1260. | ||
| + | |style="text-align:right;"|נמצא ששליש פשוט ורבע וחומש ושתות ושביעי הוא א' פשוט שלם ורל"ד חלקי' מפשוט אחד שתחלקהו לאלפי' ותק"כ חלקי‫'<br> | ||
| + | שהוא קי"ז חלקי' מאלף ור"ס וכה"ל | ||
| + | |- | ||
| + | |colspan="2"| | ||
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{3}+\frac{1}{4}+\frac{1}{5}+\frac{1}{6}+\frac{1}{7}=\frac{840+630+504+420+360}{2520}=\frac{2754}{2520}=1+\frac{234}{2520}=1+\frac{117}{1260}}}</math> | ||
|- | |- | ||
| | | | ||
| − | :{{#annot:lance|650|DcTH}}3) | + | |
| − | : | + | === <span style=color:green>Find a Quantity Problem - Whole from Parts Problem - Lance</span> === |
| + | |||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :{{#annot:lance|650|DcTH}}3) Question: one-third of the lance is in the water, its quarter is in the ground, and 10 cubits of it are up above the water. | ||
| + | :How many cubits is the whole lance? | ||
:<math>\scriptstyle\frac{1}{3}X+\frac{1}{4}X+10=X</math> | :<math>\scriptstyle\frac{1}{3}X+\frac{1}{4}X+10=X</math> | ||
| − | |style="text-align:right;"|ג | + | |style="text-align:right;"|ג <big>שאלה</big> רומח אחד שלישיתו במים ורביעיתו בעפר ולמעלה מן המים י' אמות<br> |
כמה אמות כל הרומח{{#annotend:DcTH}} | כמה אמות כל הרומח{{#annotend:DcTH}} | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''False Position:'''</span> We look for a number that has a third and a quarter; it is 12. | |
|style="text-align:right;"|נבקש מניין שיש לו שלישית ורביעית והוא י"ב | |style="text-align:right;"|נבקש מניין שיש לו שלישית ורביעית והוא י"ב | ||
|- | |- | ||
| | | | ||
| − | : | + | :The sum of its third and its quarter is 7. |
| − | |style="text-align:right;"|ושלישיתו ורביעיתו מחוברים ז | + | |style="text-align:right;"|ושלישיתו ורביעיתו מחוברים ז‫' |
|- | |- | ||
| | | | ||
| − | : | + | :We subtract it from 12; 5 remains. |
| − | : | + | :<math>\scriptstyle{\color{blue}{12-\left[\left(\frac{1}{3}\sdot12\right)+\left(\frac{1}{4}\sdot12\right)\right]=12-7=5}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|נחסרם מי"ב ישארו ה‫' |
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :<span style=color:green>'''Rule of Three:'''</span> |
| − | |style="text-align:right;"| | + | :We multiply the extremes; the result is 120. |
| + | |style="text-align:right;"|כפלנו הקצוות עלו ק"כ | ||
|- | |- | ||
| − | |||
| | | | ||
| + | :We divide it by 5; the result is 24 and this is the length of the whole lance. | ||
| + | :<math>\scriptstyle{\color{blue}{x=\frac{12\sdot10}{5}=\frac{120}{5}=24}}</math> | ||
| + | |style="text-align:right;"|חלקנום על ה' עלה כ"ד וזהו גבהות כל הרומח | ||
|- | |- | ||
| | | | ||
| − | :{{#annot:money|648|XcUw}}4) | + | :<span style=color:green>'''Check:'''</span> Its third is 8; its quarter is 6; we subtract them from 24; 10 integers remain no more and no less. |
| − | :How much is the money? | + | :<math>\scriptstyle{\color{blue}{24-\left(\frac{1}{3}\sdot24\right)-\left(\frac{1}{4}\sdot24\right)=24-8-6=10}}</math> |
| + | |style="text-align:right;"|שלישיתו ח' ורביעיתו ו' נחסרם מכ"ד ישארו י' שלמים בלי תוספת ומגרעת וכה"ל | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | === <span style=color:green>How Much Problem - Money</span> === | ||
| + | |||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :{{#annot:money|648|XcUw}}4) Question: An amount of money - we sum its fifth, its seventh, and its ninth; they are 10. | ||
| + | :How much is the amount of money? | ||
:<math>\scriptstyle\frac{1}{5}X+\frac{1}{7}X+\frac{1}{9}X=10</math> | :<math>\scriptstyle\frac{1}{5}X+\frac{1}{7}X+\frac{1}{9}X=10</math> | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ד <big>שאלה</big> ממון חברנו חמישיתו ושביעיתו ותשיעיתו והיו י‫'<br> |
כמה הממון{{#annotend:XcUw}} | כמה הממון{{#annotend:XcUw}} | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''False Position - common denominator:'''</span> We look for the denominator; it is 315 and the parts are 143. | |
|style="text-align:right;"|נבקש המורה והוא שט"ו והחלקי' ההם קמ"ג | |style="text-align:right;"|נבקש המורה והוא שט"ו והחלקי' ההם קמ"ג | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\frac{1}{5}\sdot315=63}}</math> | + | :When the part of 315 is a fifth, the result is 63. |
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{5}\sdot315=63}}</math> | ||
|style="text-align:right;"|כאשר החלק שט"ו של ה' יעלו ס"ג | |style="text-align:right;"|כאשר החלק שט"ו של ה' יעלו ס"ג | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\frac{1}{7}\sdot315=45}}</math> | + | :When the part is a seventh, the result is 45. |
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{7}\sdot315=45}}</math> | ||
|style="text-align:right;"|וכשהחלק של ז' יעלו מ"ה | |style="text-align:right;"|וכשהחלק של ז' יעלו מ"ה | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\frac{1}{9}\sdot315=35}}</math> | + | :When the part is a ninth, the result is 35. |
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{9}\sdot315=35}}</math> | ||
|style="text-align:right;"|וכשהחלק של ט' יעלו ל"ה | |style="text-align:right;"|וכשהחלק של ט' יעלו ל"ה | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{63+45+35=143}}</math> | + | :Sum them up; the result is 143. |
| + | :<math>\scriptstyle{\color{blue}{63+45+35=143}}</math> | ||
|style="text-align:right;"|{{#annot:term|178,1165|fIwp}}חברם יחד{{#annotend:fIwp}} יעלו קמ"ג | |style="text-align:right;"|{{#annot:term|178,1165|fIwp}}חברם יחד{{#annotend:fIwp}} יעלו קמ"ג | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> We multiply 315 by 10; the result is 3150. | |
| − | + | |style="text-align:right;"|נכפול שט"ו על י' עלו ג' אלפי' וק"נ | |
| − | |style="text-align:right;"|נכפול שט"ו על י' עלו ג' אלפי' וק"נ | ||
|- | |- | ||
| | | | ||
| − | : | + | :We divide it by 143; the result is 22 integers and 4 parts of 143 and this is the amount of money. |
| + | :<math>\scriptstyle{\color{blue}{x=\frac{315\sdot10}{143}=\frac{3150}{143}=22+\frac{4}{143}}}</math> | ||
| + | |style="text-align:right;"|חלקנום על קמ"ג עלו כ"ב שלמי' וד' חלקי' מן קמ"ג וככה הממון | ||
| + | |- | ||
| | | | ||
| + | :<span style=color:green>'''Check:'''</span> Take a fifth of 22; it is 4 and 2 remain that are 2 times 143, which is 286. | ||
| + | |style="text-align:right;"|קח חמישית כ"ב הם ד' נשאר ב' שהם ב' פעמי' קמ"ג והם רפ"ו | ||
|- | |- | ||
| | | | ||
| − | : | + | :Add to it 4 parts; it is 290. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|{{#annot:term|178,1165|47QY}}חבר עליהם{{#annotend:47QY}} ד' חלקי' הרי ר"צ |
| − | |||
| − | {{#annot:term|178,1165|47QY}}חבר עליהם{{#annotend:47QY}} ד' חלקי' הרי ר"צ | ||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :Divide it by 5; the result is 58 parts of 143. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|חלקם בה' יעלו נ"ח חלקי' מקמ"ג |
|- | |- | ||
| + | |colspan="2"| | ||
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{5}\sdot\left(22+\frac{4}{143}\right)=4+\frac{2}{5}+\frac{4}{5\sdot143}=4+\frac{\left(2\sdot143\right)+4}{5\sdot143}=4+\frac{286+4}{5\sdot143}=4+\frac{290}{5\sdot143}=4+\frac{58}{143}}}</math> | ||
| | | | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{315-143=172}}</math> | + | :Do likewise with the ninth and the seventh; and the result is 10. |
| + | |style="text-align:right;"|וכן עשה מן התשיעית והשבעית ויעלו י‫' | ||
| + | |- | ||
| + | |colspan="2"| | ||
| + | :<math>\scriptstyle{\color{blue}{\left[\frac{1}{5}\sdot\left(22+\frac{4}{143}\right)\right]+\left[\frac{1}{7}\sdot\left(22+\frac{4}{143}\right)\right]+\left[\frac{1}{9}\sdot\left(22+\frac{4}{143}\right)\right]=10}}</math> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :It has another method: | ||
| + | |style="text-align:right;"|עוד יש בו כלל אחר | ||
| + | |- | ||
| + | | | ||
| + | :Consider the fractions you sum that are 10 as 143 and the original amount of money as 172. | ||
| + | :<math>\scriptstyle{\color{blue}{315-143=172}}</math> | ||
|style="text-align:right;"|שתאמר החלקי' שהוספת שהם י' הם קמ"ג והממון הראשון היה קע"ב | |style="text-align:right;"|שתאמר החלקי' שהוספת שהם י' הם קמ"ג והממון הראשון היה קע"ב | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> say: if 143 equals 10, how much is 172 equal to? | |
| + | :<math>\scriptstyle{\color{blue}{143:10=172:a}}</math> | ||
|style="text-align:right;"|אמור אם קמ"ג שוים י' קע"ב כמה שוים | |style="text-align:right;"|אמור אם קמ"ג שוים י' קע"ב כמה שוים | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{a=\frac{10\sdot172}{143}=\frac{1720}{143}=12+\frac{4}{143}}}</math> | + | :Say: 10 times 172 is 1720. Divide it by 143; the result is 12 integers and 4 parts of 143. |
| − | |style="text-align:right;"|אמור י' פעמי' קע"ב הם אלף | + | :<math>\scriptstyle{\color{blue}{a=\frac{10\sdot172}{143}=\frac{1720}{143}=12+\frac{4}{143}}}</math> |
| + | |style="text-align:right;"|אמור י' פעמי' קע"ב הם אלף תש"כ<br> | ||
| + | חלקם על קמ"ג יבאו י"ב שלמי' וד' חלקי' מקמ"ג | ||
|- | |- | ||
| | | | ||
| − | : | + | :Add the 10 to it; it is 22 integers and 4 parts of 143 no more and no less. |
| + | :<math>\scriptstyle{\color{blue}{x=\left(12+\frac{4}{143}\right)+10=22+\frac{4}{143}}}</math> | ||
|style="text-align:right;"|חבר אליהם הי' יהיו כ"ב שלמי' וד' חלקי' מקמ"ג בלי תוספת ומגרעת | |style="text-align:right;"|חבר אליהם הי' יהיו כ"ב שלמי' וד' חלקי' מקמ"ג בלי תוספת ומגרעת | ||
|- | |- | ||
| | | | ||
| + | :This rule is also suitable for knowing the measure of the lance mentioned above. | ||
|style="text-align:right;"|וכן כ.. מה הכלל ג"כ הוא טוב לדעת מדת הרומח הכתוב למעלה | |style="text-align:right;"|וכן כ.. מה הכלל ג"כ הוא טוב לדעת מדת הרומח הכתוב למעלה | ||
|- | |- | ||
| − | | | + | | |
| + | |||
| + | === <span style=color:green>Find a Quantity Problem - First from Last Problem – Money</span> === | ||
| + | |||
| | | | ||
|- | |- | ||
| | | | ||
| − | :{{#annot:money|651|AtgS}}5) An amount of money - its fifth, its seventh, and its ninth | + | :{{#annot:money|651|AtgS}}5) We do the opposite: An amount of money - we subtract its fifth, its seventh, and its ninth from it and 10 remains. |
:<math>\scriptstyle X-\left(\frac{1}{5}X+\frac{1}{7}X+\frac{1}{9}X\right)=10</math> | :<math>\scriptstyle X-\left(\frac{1}{5}X+\frac{1}{7}X+\frac{1}{9}X\right)=10</math> | ||
| − | |style="text-align:right;"|ה | + | |style="text-align:right;"|‫<ref>88v</ref>ה <big>נעשה</big> להפך ממון חסרנו ממנו חמשיתו ושבעיתו ותשיעיתו ונשארו י‫'{{#annotend:AtgS}} |
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{315-143=172}}</math> | + | :We subtract 143, which are the fractions, from 315, which is the denominator; 172 remains. |
| + | :<math>\scriptstyle{\color{blue}{315-143=172}}</math> | ||
|style="text-align:right;"|נחסר קמ"ג שהם השברי' משט"ו שהוא המורה ישאר קע"ב | |style="text-align:right;"|נחסר קמ"ג שהם השברי' משט"ו שהוא המורה ישאר קע"ב | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> we do as follows: we multiply 10 by 315; the result is 3150. We divide it by 172; the result is 18 integers and 54 parts of 172. | |
| − | + | :<math>\scriptstyle{\color{blue}{x=\frac{10\sdot315}{172}=\frac{3150}{172}=18+\frac{54}{172}}}</math> | |
| − | |style="text-align:right;"|ונעשה כך כפלנו י' על שט"ו עלו ג' אלפי' וק"נ חלקנום על קע"ב עלו י"ח שלמי' ונ"ד חלקי' מקע"ב | + | |style="text-align:right;"|ונעשה כך כפלנו י' על שט"ו עלו ג' אלפי' וק"נ<br> |
| + | חלקנום על קע"ב עלו י"ח שלמי' ונ"ד חלקי' מקע"ב | ||
|- | |- | ||
| | | | ||
| − | :Check: | + | :<span style=color:green>'''Check:'''</span> we take a fifth, a seventh, and a ninth of this number; 10 integers remain. |
| + | |style="text-align:right;"|לקחנו חמשית ושבעית ותשעית של זה המספר ישאר י' שלמי‫' | ||
| + | |- | ||
| + | |colspan="2"| | ||
:<math>\scriptstyle{\color{blue}{\left(18+\frac{54}{172}\right)-\left[\left[\frac{1}{5}\sdot\left(18+\frac{54}{172}\right)\right]+\left[\frac{1}{7}\sdot\left(18+\frac{54}{172}\right)\right]+\left[\frac{1}{9}\sdot\left(18+\frac{54}{172}\right)\right]\right]=10}}</math> | :<math>\scriptstyle{\color{blue}{\left(18+\frac{54}{172}\right)-\left[\left[\frac{1}{5}\sdot\left(18+\frac{54}{172}\right)\right]+\left[\frac{1}{7}\sdot\left(18+\frac{54}{172}\right)\right]+\left[\frac{1}{9}\sdot\left(18+\frac{54}{172}\right)\right]\right]=10}}</math> | ||
| − | |||
|- | |- | ||
| − | | | + | | |
| + | |||
| + | === <span style=color:green>How Much Problem – Money</span> === | ||
| + | |||
| | | | ||
|- | |- | ||
| | | | ||
| − | :{{#annot:money|648|E59q}}6) An amount of money – we add to it its half, its third, its fifth, and its sixth, and the total sum is 40. | + | :{{#annot:money|648|E59q}}6) Question: An amount of money – we add to it its half, its third, its fifth, and its sixth, and the total sum is 40. |
:How much is the money? | :How much is the money? | ||
:<math>\scriptstyle X+\frac{1}{2}X+\frac{1}{3}X+\frac{1}{5}X+\frac{1}{6}X=40</math> | :<math>\scriptstyle X+\frac{1}{2}X+\frac{1}{3}X+\frac{1}{5}X+\frac{1}{6}X=40</math> | ||
| − | |style="text-align:right;"|ו | + | |style="text-align:right;"|ו <big>שאלה</big> ממון הוספנו עליו מחציתו ושלישיתו וחמישיתו וששיתו ובסך הכל היה מ‫'<br> |
כמה היה הממון{{#annotend:E59q}} | כמה היה הממון{{#annotend:E59q}} | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''False Position:'''</span> we know that a half, a third, and a sixth are one integer. We consider [the amount of money] as one; so they are two plus a fifth. | |
| − | |||
|style="text-align:right;"|ידענו כי החצי והשלישית והששית הוא אחד שלם ונחשוב כי היה אלו אחד הרי שנים יש לו תוספת החמישית | |style="text-align:right;"|ידענו כי החצי והשלישית והששית הוא אחד שלם ונחשוב כי היה אלו אחד הרי שנים יש לו תוספת החמישית | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | :<math>\scriptstyle{\color{blue}{1+\frac{1}{2}+\frac{1}{3}+\frac{1}{5}+\frac{1}{6}=1+\left(\frac{1}{2}+\frac{1}{3}+\frac{1}{6}\right)+\frac{1}{5}=1+1+\frac{1}{5}=2+\frac{1}{5}}}</math> | ||
|- | |- | ||
| | | | ||
| − | : | + | :Now, we have to divide 40 by 2 and a fifth and the result is the amount of money: we take 5 for each of the integers and add the fifth to them; they are [11]. We also multiply 40 by 5; it is 200. We divide it by 11; the result is 18 integers and 2 parts of 11. |
| − | + | :<math>\scriptstyle{\color{blue}{x=\frac{40}{2+\frac{1}{5}}=\frac{40}{\frac{\left(5\sdot2\right)+1}{5}}=\frac{40\sdot5}{11}=\frac{200}{11}=18+\frac{2}{11}}}</math> | |
|style="text-align:right;"|עתה יש לנו לחלק מ' על ב' וחמישית והעולה הוא הממון<br> | |style="text-align:right;"|עתה יש לנו לחלק מ' על ב' וחמישית והעולה הוא הממון<br> | ||
| − | הנה נקח לכל אחד מן השלמים ה' ו{{#annot:term|178,1899|pmlr}}נשים עמהם{{#annotend:pmlr}} החמשית יהיו | + | הנה נקח לכל אחד מן השלמים ה' ו{{#annot:term|178,1899|pmlr}}נשים עמהם{{#annotend:pmlr}} החמשית יהיו אלף<br> |
גם נכפול המ' על ה' עד שיהיו דרך אחד יהיו ר‫'<br> | גם נכפול המ' על ה' עד שיהיו דרך אחד יהיו ר‫'<br> | ||
נחלקנו על י"א יעלו י"ח שלמים ועוד ב' חלקי' מי"א | נחלקנו על י"א יעלו י"ח שלמים ועוד ב' חלקי' מי"א | ||
|- | |- | ||
| − | | | + | | |
| + | |||
| + | === <span style=color:green>How Much Problem – Money</span> === | ||
| + | |||
| | | | ||
|- | |- | ||
| | | | ||
| − | :{{#annot:money|648|WP7X}}7) We take a fifth amount of money, its seventh, and its ninth. | + | :{{#annot:money|648|WP7X}}7) Question: We take a fifth amount of money, its seventh, and its ninth. |
:How much is [their sum] in relation to the [original] amount of money? | :How much is [their sum] in relation to the [original] amount of money? | ||
:<math>\scriptstyle\frac{1}{5}X+\frac{1}{7}X+\frac{1}{9}X</math> | :<math>\scriptstyle\frac{1}{5}X+\frac{1}{7}X+\frac{1}{9}X</math> | ||
| − | |style="text-align:right;"|ז | + | |style="text-align:right;"|ז <big>שאלה</big> לקחנו חמישית ממון גם שביעיתו ותשיעיתו<br> |
כמה הוא מערך הממון{{#annotend:WP7X}} | כמה הוא מערך הממון{{#annotend:WP7X}} | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''False Position - common denominator:'''</span> we look for the common denominator; it is 315. | |
|style="text-align:right;"|נבקש המורה והוא שט"ו | |style="text-align:right;"|נבקש המורה והוא שט"ו | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\left(\frac{1}{5}\sdot315\right)+\left(\frac{1}{7}\sdot315\right)+\left(\frac{1}{9}\sdot315\right)=143}}</math> | + | :We sum [its] fifth, seventh, and ninth; they are 143. |
| + | :<math>\scriptstyle{\color{blue}{\left(\frac{1}{5}\sdot315\right)+\left(\frac{1}{7}\sdot315\right)+\left(\frac{1}{9}\sdot315\right)=143}}</math> | ||
|style="text-align:right;"|ונחבר חמישית ושביעית ותשיעית יהיו קמ"ג | |style="text-align:right;"|ונחבר חמישית ושביעית ותשיעית יהיו קמ"ג | ||
|- | |- | ||
| | | | ||
| − | : | + | :We divide it by 35; it is 4-ninths and 3 parts of 35 remain, because 35 is the ninth, and 5 is a seventh of the ninth, so 3 parts are 3 fifths of a seventh of a ninth. |
|style="text-align:right;"|נחלקה על ל"ה והנה הם ד' תשעיות ונשארו ג' חלקי' מל"ה<br> | |style="text-align:right;"|נחלקה על ל"ה והנה הם ד' תשעיות ונשארו ג' חלקי' מל"ה<br> | ||
כי ל"ה הוא התשיעית וה' הוא שביעית התשיעית<br> | כי ל"ה הוא התשיעית וה' הוא שביעית התשיעית<br> | ||
לכן ג' חלקי' הם ג' חמשיות שבעית התשיעית | לכן ג' חלקי' הם ג' חמשיות שבעית התשיעית | ||
|- | |- | ||
| − | | | + | | colspan="2"| |
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{5}X+\frac{1}{7}X+\frac{1}{9}X=\frac{143}{315}=\frac{\frac{143}{35}}{9}=\frac{4}{9}+\frac{\frac{3}{35}}{9}=\frac{4}{9}+\left(\frac{3}{5}\sdot\frac{1}{7}\sdot\frac{1}{9}\right)}}</math> | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | === <span style=color:green>Pricing Problem - Find the Amount</span> === | ||
| + | |||
| | | | ||
|- | |- | ||
| | | | ||
| − | :{{#annot: | + | :{{#annot:measures|630|45XD}}8) Question: A man sells 13 measures for 23. |
:How many measures will he sell for 7 [pešiṭim]? | :How many measures will he sell for 7 [pešiṭim]? | ||
:<math>\scriptstyle\frac{13}{23}=\frac{X}{7}</math> | :<math>\scriptstyle\frac{13}{23}=\frac{X}{7}</math> | ||
| − | |style="text-align:right;"|ח | + | |style="text-align:right;"|ח <big>שאלה</big> אדם מוכר י"ג מדות בכ"ג<br> |
כמה מדות יתן בז' פשוטי‫'{{#annotend:45XD}} | כמה מדות יתן בז' פשוטי‫'{{#annotend:45XD}} | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> we say: 7 times 13 is 91. We divide it by 23; the result is 3 measures and 22 of 23 parts of one measure. | |
| + | :<math>\scriptstyle{\color{blue}{x=\frac{7\sdot13}{23}=\frac{91}{23}=3+\frac{22}{23}}}</math> | ||
|style="text-align:right;"|נאמר ז' פעמי' י"ג יהיו צ"א<br> | |style="text-align:right;"|נאמר ז' פעמי' י"ג יהיו צ"א<br> | ||
נחלקם על כ"ג בא ג' מדות וכ"ב מכ"ג חלקי' במדה אחת | נחלקם על כ"ג בא ג' מדות וכ"ב מכ"ג חלקי' במדה אחת | ||
|- | |- | ||
| − | | | + | | |
| + | |||
| + | === <span style=color:green>Pricing Problem - Find the Price</span> === | ||
| + | |||
| | | | ||
|- | |- | ||
| | | | ||
| − | * | + | *Now, we reverse the calculation and want to know how much will he get for 7 measures? |
:<math>\scriptstyle\frac{13}{23}=\frac{7}{X}</math> | :<math>\scriptstyle\frac{13}{23}=\frac{7}{X}</math> | ||
|style="text-align:right;"|ועת' נהפוך חשבון שנבקש לדעת בכמה יתן לו ז' מדות | |style="text-align:right;"|ועת' נהפוך חשבון שנבקש לדעת בכמה יתן לו ז' מדות | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> we say: 7 times 23 is 161. We divide it by 17; it is 12 and 5 parts of 13 of one pašuṭ. | |
| + | :<math>\scriptstyle{\color{blue}{x=\frac{7\sdot23}{17}=\frac{161}{17}=12+\frac{5}{13}}}</math> | ||
|style="text-align:right;"|נאמר ז' פעמים כ"ג הם קס"א<br> | |style="text-align:right;"|נאמר ז' פעמים כ"ג הם קס"א<br> | ||
נחלקם על י"ז יהיו י"ב וה' חלקי' מי"ג בפשוט | נחלקם על י"ז יהיו י"ב וה' חלקי' מי"ג בפשוט | ||
|- | |- | ||
| − | | | + | | |
| + | |||
| + | === <span style=color:green>Exchange Problem - Currencies</span> === | ||
| + | |||
| | | | ||
|- | |- | ||
| Line 314: | Line 435: | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> <math>\scriptstyle{\color{blue}{x=\frac{5\sdot4}{3}=\frac{20}{3}=6+\frac{2}{3}}}</math> of Cortona | |
|style="text-align:right;"|אמור ה' פעמי' ד' הם כ‫'<br> | |style="text-align:right;"|אמור ה' פעמי' ד' הם כ‫'<br> | ||
| − | חלקם על ג' בא ו' קורטוניס וב' שלשי' | + | חלקם על ג' בא ו' קורטוניס וב' שלשי' וכה"ל |
|- | |- | ||
| − | | | + | | |
| + | === <span style=color:green>Motion Problem – Pursuit</span> === | ||
| + | |||
| | | | ||
|- | |- | ||
| | | | ||
| − | :{{#annot:messenger|657|yABa}}9) A man sent a messenger to walk 29 miles a day. | + | :{{#annot:messenger|657|yABa}}9) Question: A man sent a messenger to walk 29 miles a day. |
:After 10 days of walking, he sent another messenger to walk after him 37 miles a day. | :After 10 days of walking, he sent another messenger to walk after him 37 miles a day. | ||
:When will he catch up with him?<br> | :When will he catch up with him?<br> | ||
:<math>\scriptstyle29X=37\sdot\left(X-10\right)</math> | :<math>\scriptstyle29X=37\sdot\left(X-10\right)</math> | ||
| − | |style="text-align:right;"|ט | + | |style="text-align:right;"|ט <big>שאלה</big> אדם שלח רץ שילך בכל יום כ"ט מילים<br> |
אחר מהלך י' ימי' שלח רץ אחר אחריו שילך בכל יום ל"ז מילי‫'<br> | אחר מהלך י' ימי' שלח רץ אחר אחריו שילך בכל יום ל"ז מילי‫'<br> | ||
מתי ישיגנו{{#annotend:yABa}} | מתי ישיגנו{{#annotend:yABa}} | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> we multiply the miles he walks by 10 days; it is 290. We divide it by the difference between the two velocities, which is 8; it is 36 days and a quarter of a day. | |
| + | :<math>\scriptstyle{\color{blue}{x=\frac{29\sdot10}{37-29}=\frac{290}{8}=36+\frac{1}{4}}}</math> | ||
|style="text-align:right;"|נכפול המילים שהלך בי' ימי' יהיו ר"צ<br> | |style="text-align:right;"|נכפול המילים שהלך בי' ימי' יהיו ר"צ<br> | ||
נחלקם על היתרון שבין שני המהלכים שהוא ח' והנו ל"ו ימים ורבע יום | נחלקם על היתרון שבין שני המהלכים שהוא ח' והנו ל"ו ימים ורבע יום | ||
|- | |- | ||
| − | | | + | | |
| + | |||
| + | === <span style=color:green>Motion Problem – Encounter</span> === | ||
| + | |||
| | | | ||
|- | |- | ||
| | | | ||
| − | :{{#annot:brothers|658|nkb8}}10) Reuven left his city, walking to Shimon, to his city, on Sunday morning of the first of the month. | + | :{{#annot:brothers|658|nkb8}}10) Question: Reuven left his city, walking to Shimon, to his city, on Sunday morning of the first of the month. |
:Shimon left his city on that same day, walking to Reuven's city. | :Shimon left his city on that same day, walking to Reuven's city. | ||
:The distance between the two cities is 100 miles. | :The distance between the two cities is 100 miles. | ||
| Line 345: | Line 472: | ||
:When will they meet? | :When will they meet? | ||
:<math>\scriptstyle19X+17X=100</math> | :<math>\scriptstyle19X+17X=100</math> | ||
| − | |style="text-align:right;"|י | + | |style="text-align:right;"|י <big>שאלה</big> ראובן יצא מעירו ללכת לקראת שמעון לעירו בקר יום ראשון של ר"ח<br> |
ובאותו יום עצמו יצא שמעון מעירו ללכת לעיר ראובן<br> | ובאותו יום עצמו יצא שמעון מעירו ללכת לעיר ראובן<br> | ||
והמרחק בין שני הערים ק' מלים<br> | והמרחק בין שני הערים ק' מלים<br> | ||
| Line 352: | Line 479: | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{x=\frac{100}{19+17}=\frac{100}{36}=2+\frac{28}{36}=2+\frac{7}{9}}}</math> | + | :Do as follows: sum up the two velocities; it is 36. Divide the 100 miles by it; it is 2 days and 28 parts of 36 of one day remain, which are 7-ninths of a day. |
| + | :<math>\scriptstyle{\color{blue}{x=\frac{100}{19+17}=\frac{100}{36}=2+\frac{28}{36}=2+\frac{7}{9}}}</math> | ||
|style="text-align:right;"|ככה תעשה חבר שני המהלכי' הם ל"ו<br> | |style="text-align:right;"|ככה תעשה חבר שני המהלכי' הם ל"ו<br> | ||
חלק עליו הק' מילים יהיו ב' ימים ישארו כ"ח חלקי' מל"ו ביום אחד שהם ז' תשעיו' יום | חלק עליו הק' מילים יהיו ב' ימים ישארו כ"ח חלקי' מל"ו ביום אחד שהם ז' תשעיו' יום | ||
|- | |- | ||
| − | | | + | | |
| + | |||
| + | === <span style=color:green>How Many Problem - Group of People</span> === | ||
| + | |||
| | | | ||
|- | |- | ||
| | | | ||
| − | :{{#annot:group of people|649|5A21}}11) A man passed by a group of people. | + | :{{#annot:group of people|649|5A21}}11) Question: A man passed by a group of people. |
:He said to them: hello one hundred people. | :He said to them: hello one hundred people. | ||
:They answered him: we are not one hundred people, but all of us, and other like us, and half of us, and a quarter of us plus one will make 100 | :They answered him: we are not one hundred people, but all of us, and other like us, and half of us, and a quarter of us plus one will make 100 | ||
:<math>\scriptstyle X+X+\frac{1}{2}X+\frac{1}{4}X+1=100</math> | :<math>\scriptstyle X+X+\frac{1}{2}X+\frac{1}{4}X+1=100</math> | ||
| − | |style="text-align:right;"|יא | + | |style="text-align:right;"|יא <big>שאלה</big> אדם עבר על אנשים<br> |
אמר להם שלו' לכם מאה איש<br> | אמר להם שלו' לכם מאה איש<br> | ||
ענו לו אין אנו מאה רק אנו ואחרים כמונו ומחציתנו ורביעתנו עדיף נהיה מאה{{#annotend:5A21}} | ענו לו אין אנו מאה רק אנו ואחרים כמונו ומחציתנו ורביעתנו עדיף נהיה מאה{{#annotend:5A21}} | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''False Position:'''</span> we take one for their number and one "like us" - it is two; and "half of us" - it is two and a half. We add a quarter; it is [2 and] 3-quarters. | |
| − | + | |style="text-align:right;"|והנה נקח למספרם אחד ואחד כמונו והנה שנים ומחציתנו חצי אחד הנה שנים וחצי<br> | |
| − | |style="text-align:right;"|והנה נקח למספרם אחד ואחד כמונו והנה שנים | + | נוסיף רבעיתו יהיו ‫<ref>89r</ref>ג' רבעיות |
| − | ומחציתנו חצי אחד הנה שנים וחצי<br> | ||
| − | נוסיף רבעיתו יהיו | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :Since we have quarter[s], we take 4 for each unit; they are 8. We add the 3-quarters to them; they are 11. |
| − | + | |style="text-align:right;"|ובעבור שיש לנו רבעית נקח לכל שלם ד' ויהיו ח‫'<br> | |
| − | |style="text-align:right;"|ובעבור שאמרו שיהיו עמו מאה יהיה מספרם עם התוספת צ"ט | + | נחבר אליהם הג' רבעים יהיו י"א |
| − | נשיבם מהדרך הד' יהיו שצ"ו | + | |- |
| − | + | | colspan="2"| | |
| + | :<math>\scriptstyle{\color{blue}{1+1+\frac{1}{2}+\frac{1}{4}=2+\frac{1}{2}+\frac{1}{4}=2+\frac{3}{4}=\frac{\left(4\sdot2\right)+3}{4}=\frac{8+3}{4}=\frac{11}{4}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :Since they said that they are one hundred with him, their number with the additions is 99. | ||
| + | |style="text-align:right;"|ובעבור שאמרו שיהיו עמו מאה יהיה מספרם עם התוספת צ"ט | ||
| + | |- | ||
| + | | | ||
| + | :We convert them into quarters; they are 396. | ||
| + | |style="text-align:right;"|נשיבם מהדרך הד' יהיו שצ"ו | ||
|- | |- | ||
| − | |||
| | | | ||
| + | :We divide them by 11; they are 36 and this is their number. | ||
| + | :<math>\scriptstyle{\color{blue}{x=\frac{4\sdot\left(100-1\right)}{11}=\frac{4\sdot99}{11}=\frac{396}{11}=36}}</math> | ||
| + | |style="text-align:right;"|נחלקם על י"א יהיו ל"ו וככה מספרם | ||
|- | |- | ||
| | | | ||
| − | :{{#annot:WP|641|cW4Z}}12) A man bought 100 liṭra for 100 zehuvim. | + | |
| − | :He sold 50 [of them] at | + | === <span style=color:green>Buy and Sell Problem</span> === |
| + | |||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :{{#annot:WP|641|cW4Z}}12) Question: A man bought 100 liṭra for 100 zehuvim. | ||
| + | :He sold 50 [of them] at a liṭra and a quarter for one zahuv, and the other 50 at a liṭra minus a quarter for one zahuv. | ||
:We want to know: did he earn or lose? | :We want to know: did he earn or lose? | ||
:<math>\scriptstyle\left(\frac{50}{1+\frac{1}{4}}+\frac{50}{1-\frac{1}{4}}\right)-100</math> | :<math>\scriptstyle\left(\frac{50}{1+\frac{1}{4}}+\frac{50}{1-\frac{1}{4}}\right)-100</math> | ||
| − | |style="text-align:right;"|יב | + | |style="text-align:right;"|יב <big>שאלה</big> אדם קנה בק' זהובים ק' ליט‫'<br> |
אחרי כן מכר הנ' ליט' ורבע ליט' בזהוב והנ' ליט' מכר ליט' פחות רבע ליט' בזהוב<br> | אחרי כן מכר הנ' ליט' ורבע ליט' בזהוב והנ' ליט' מכר ליט' פחות רבע ליט' בזהוב<br> | ||
נבקש לדעת אם הרויח או הפסיד{{#annotend:cW4Z}} | נבקש לדעת אם הרויח או הפסיד{{#annotend:cW4Z}} | ||
|- | |- | ||
| | | | ||
| − | : | + | :We convert the first 50 into 200, because they are quarters, then divide them by 5, as he sold a liṭra and a quarter for one zahuv; they are 40 zehuvim. |
| − | + | :<math>\scriptstyle{\color{blue}{\frac{50}{1+\frac{1}{4}}=\frac{4\sdot50}{5}=\frac{200}{5}=40}}</math> | |
|style="text-align:right;"|נשיב הנ' ראשונים ר' כי רבעים הם<br> | |style="text-align:right;"|נשיב הנ' ראשונים ר' כי רבעים הם<br> | ||
נחלקם על ה' כי ליט' ורבע ליט' מכר בזהוב יהיו מ' זהובי‫' | נחלקם על ה' כי ליט' ורבע ליט' מכר בזהוב יהיו מ' זהובי‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | :We multiply the other 50 by 4 also; they are 200. We divide them by 3, as he sold 3-quarters for one zahuv; they are 66 zehuvim and two-thirds of a zahuv. |
| − | + | :<math>\scriptstyle{\color{blue}{\frac{50}{1-\frac{1}{4}}=\frac{4\sdot50}{3}=\frac{200}{3}=66+\frac{2}{3}}}</math> | |
|style="text-align:right;"|גם נכפול הנ' אחרים על ד' יהיו ר‫'<br> | |style="text-align:right;"|גם נכפול הנ' אחרים על ד' יהיו ר‫'<br> | ||
נחלקם על ג' כי ג' רבעים מכר בזהוב והנה יהיו ס"ו זהובים ושני שלישי זהוב | נחלקם על ג' כי ג' רבעים מכר בזהוב והנה יהיו ס"ו זהובים ושני שלישי זהוב | ||
|- | |- | ||
| | | | ||
| − | : | + | :Add the 40 to them; the profit is 6 zehuvim and two-thirds of a zahuv. |
| − | + | |style="text-align:right;"|וחבר אליהם המ' יהיה הריוח ו' זהובים ושני שלישי זהוב וכה"ל | |
| − | + | |- | |
| + | | colspan="2"| | ||
| + | :<math>\scriptstyle{\color{blue}{\left(\frac{50}{1+\frac{1}{4}}+\frac{50}{1-\frac{1}{4}}\right)-100=\left[40+\left(66+\frac{2}{3}\right)\right]-100=6+\frac{2}{3}}}</math> | ||
|- | |- | ||
| − | | | + | | |
| + | |||
| + | === <span style=color:green>Boiling Problem</span> === | ||
| + | |||
| | | | ||
|- | |- | ||
| | | | ||
| − | :{{#annot:WP|663|tVUC}}13) A man had 10 measures of apricot and he wanted to boil them so that only one third will remain. | + | :{{#annot:WP|663|tVUC}}13) Question: A man had 10 measures of apricot and he wanted to boil them so that only one third will remain. |
:He started to cook [them] until eight measures remained of them. | :He started to cook [them] until eight measures remained of them. | ||
:Then two measures overflow. | :Then two measures overflow. | ||
:Now he wants to boil [the remainder] until it will be reduced [as planned for] the original [amount] of apricot | :Now he wants to boil [the remainder] until it will be reduced [as planned for] the original [amount] of apricot | ||
:<math>\scriptstyle\frac{8}{\frac{1}{3}\sdot10}=\frac{8-2}{X}</math> | :<math>\scriptstyle\frac{8}{\frac{1}{3}\sdot10}=\frac{8-2}{X}</math> | ||
| − | |style="text-align:right;"|יג | + | |style="text-align:right;"|יג <big>שאלה</big> אדם היו לו י' מדות משמש ורוצה לבשלם עד שלא ישאר כי אם השלישית<br> |
והנה החל לבשל עד שנשארו מהם ח' מדות<br> | והנה החל לבשל עד שנשארו מהם ח' מדות<br> | ||
ונשפך מהם ב' מדות<br> | ונשפך מהם ב' מדות<br> | ||
| Line 428: | Line 577: | ||
|- | |- | ||
| | | | ||
| − | : | + | :You have three known numbers: |
|style="text-align:right;"|ועתה יש לך ג' מספרים ידועים | |style="text-align:right;"|ועתה יש לך ג' מספרים ידועים | ||
|- | |- | ||
| | | | ||
| − | :: | + | :The first is a third of 10, which is 3 and a third. |
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{3}\sdot10=3+\frac{1}{3}}}</math> | ||
|style="text-align:right;"|האחד שליש י' והוא ג' ושליש | |style="text-align:right;"|האחד שליש י' והוא ג' ושליש | ||
|- | |- | ||
| | | | ||
| − | : | + | :The second is the 8 cooked measures. |
|style="text-align:right;"|והשני ח' הם המדות שנתבשלו | |style="text-align:right;"|והשני ח' הם המדות שנתבשלו | ||
|- | |- | ||
| | | | ||
| − | : | + | :The third is the 6 [measures] left over from what was overflowed. |
|style="text-align:right;"|והשלישי ו' שנשארו מן הנשפך | |style="text-align:right;"|והשלישי ו' שנשארו מן הנשפך | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> we multiply 6 by 3 and a third; it is 20. Divide it by 8; it is 2 and a half. | |
| + | :<math>\scriptstyle{\color{blue}{x=\frac{\left(3+\frac{1}{3}\right)\sdot6}{8}=\frac{20}{8}=2+\frac{1}{2}}}</math> | ||
|style="text-align:right;"|והנה נכפול ו' על ג' ושליש יהיו כ‫'<br> | |style="text-align:right;"|והנה נכפול ו' על ג' ושליש יהיו כ‫'<br> | ||
| − | וחלקם על ח' יהיו ב וחצי | + | וחלקם על ח' יהיו ב' וחצי |
|- | |- | ||
| − | | | + | | |
| + | |||
| + | === <span style=color:green>Partnership Problem - For the Same Time</span> === | ||
| + | |||
| | | | ||
|- | |- | ||
| | | | ||
| − | :{{#annot:four partners|661|4fUT}}14) Four people: one of them had 11 dinar, the second had 13 dinar, the third had 15 dinar, and the fourth had 17 dinar. | + | :{{#annot:four partners|661|4fUT}}14) Question: Four people: one of them had 11 dinar, the second had 13 dinar, the third had 15 dinar, and the fourth had 17 dinar. |
:They earned 19 dinar. | :They earned 19 dinar. | ||
:How much should each of them take [from the profit]? | :How much should each of them take [from the profit]? | ||
| − | |style="text-align:right;"|יד | + | |style="text-align:right;"|יד <big>שאלה</big> ד' אנשים יש לאחד מהם י"א דינרי' ולשני י"ג די' ולשלישי ט"ו דינרי' ולרבעי י"ז דינרי‫'<br> |
והרויחו י"ט דינרי‫'<br> | והרויחו י"ט דינרי‫'<br> | ||
כמה יקח כל אחד ואחד{{#annotend:4fUT}} | כמה יקח כל אחד ואחד{{#annotend:4fUT}} | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''False Position:'''</span> we sum up all four amounts of their money; it is 56. | |
| + | :<math>\scriptstyle{\color{blue}{11+13+15+17=56}}</math> | ||
|style="text-align:right;"|נחבר ראשי' כל ד' ממונם ויהיו נ"ו | |style="text-align:right;"|נחבר ראשי' כל ד' ממונם ויהיו נ"ו | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> as the ratio of each to 56, so each takes from 19. | |
| + | :<math>\scriptstyle{\color{blue}{\frac{a_i}{56}=\frac{x_i}{19}}}</math> | ||
|style="text-align:right;"|ובערך כל אחד אל נ"ו ככה יקח מי"ט | |style="text-align:right;"|ובערך כל אחד אל נ"ו ככה יקח מי"ט | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{x_1=\frac{11\sdot19}{56}=\frac{209}{56}=3+\frac{41}{56}}}</math> | + | :We do as follows: we multiply 11 by 19; the result is 209. We divide [it] by 56; the result is 3 integers and 41 parts. |
| + | :<math>\scriptstyle{\color{blue}{x_1=\frac{11\sdot19}{56}=\frac{209}{56}=3+\frac{41}{56}}}</math> | ||
|style="text-align:right;"|ונעשה כך נכפול י"א על י"ט יעלו ר"ט<br> | |style="text-align:right;"|ונעשה כך נכפול י"א על י"ט יעלו ר"ט<br> | ||
נחלק על נ"ו יעלו ג' שלמי' ומ"א חלקי‫' | נחלק על נ"ו יעלו ג' שלמי' ומ"א חלקי‫' | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{x_2=\frac{13\sdot19}{56}=\frac{247}{56}=4+\frac{23}{56}}}</math> | + | :We do the same with 13; the result is 247. We divide it by 56; the result is 4 integers and 23 parts. |
| + | :<math>\scriptstyle{\color{blue}{x_2=\frac{13\sdot19}{56}=\frac{247}{56}=4+\frac{23}{56}}}</math> | ||
|style="text-align:right;"|עשינו כן בי"ג עלו רמ"ז<br> | |style="text-align:right;"|עשינו כן בי"ג עלו רמ"ז<br> | ||
חלקנום על נ"ו עלו ד' שלמי' וכ"ג חלקי‫' | חלקנום על נ"ו עלו ד' שלמי' וכ"ג חלקי‫' | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{x_3=\frac{15\sdot19}{56}=\frac{285}{56}=5+\frac{5}{56}}}</math> | + | :We do the same with 15; it is 285. We divide it by 56; the result is 5 integers and 5 parts. |
| + | :<math>\scriptstyle{\color{blue}{x_3=\frac{15\sdot19}{56}=\frac{285}{56}=5+\frac{5}{56}}}</math> | ||
|style="text-align:right;"|עשינו כן בט"ו יהיו רפ"ה<br> | |style="text-align:right;"|עשינו כן בט"ו יהיו רפ"ה<br> | ||
חלקנום על נ"ו עלו ה' שלמי' וה' חלקי‫' | חלקנום על נ"ו עלו ה' שלמי' וה' חלקי‫' | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{x_4=\frac{17\sdot19}{56}=\frac{323}{56}=5+\frac{43}{56}}}</math> | + | :We do the same with 17; the result is 323. We divide it by 56; the result is 5 integers and 43 parts. |
| + | :<math>\scriptstyle{\color{blue}{x_4=\frac{17\sdot19}{56}=\frac{323}{56}=5+\frac{43}{56}}}</math> | ||
|style="text-align:right;"|עשינו כן בי"ז עלו שכ"ג<br> | |style="text-align:right;"|עשינו כן בי"ז עלו שכ"ג<br> | ||
חלקנום על נ"ו עלו ה' שלמי' ומ"ג חלקי‫' | חלקנום על נ"ו עלו ה' שלמי' ומ"ג חלקי‫' | ||
|- | |- | ||
| | | | ||
| − | :Check: <math>\scriptstyle{\color{blue}{\left(3+\frac{41}{56}\right)+\left(4+\frac{23}{56}\right)+\left(5+\frac{5}{56}\right)+\left(5+\frac{43}{56}\right)=19}}</math> | + | :<span style=color:green>'''Check:'''</span> we sum up the integers and the fractions; the result is 19 integers, because the fractions are 56 parts. |
| + | :<math>\scriptstyle{\color{blue}{\left(3+\frac{41}{56}\right)+\left(4+\frac{23}{56}\right)+\left(5+\frac{5}{56}\right)+\left(5+\frac{43}{56}\right)=19}}</math> | ||
|style="text-align:right;"|חברנו אלו השלמי' ואלו החלקי' עלו י"ט שלמי' כי החלקי' האלו חלקי נ"ו הם | |style="text-align:right;"|חברנו אלו השלמי' ואלו החלקי' עלו י"ט שלמי' כי החלקי' האלו חלקי נ"ו הם | ||
|- | |- | ||
| − | | | + | | |
| + | |||
| + | === <span style=color:green>Purchase Problem – Moneychanger</span> === | ||
| + | |||
| | | | ||
|- | |- | ||
| | | | ||
| − | :{{#annot:moneychanger|642|JBea}}15) The moneychanger has three [kinds of] coins. | + | :{{#annot:moneychanger|642|JBea}}15) Question: The moneychanger has three [kinds of] coins. |
:One zahuv is worth three dinar of the first [kind of] coins; or four of the second [kind]; or six of the third [kind]. | :One zahuv is worth three dinar of the first [kind of] coins; or four of the second [kind]; or six of the third [kind]. | ||
:A man came and asked the moneychanger to give him from the three [kinds of] coins for one zahuv equally, so that the amount of the expensive will be equal to the amount of the inexpensive. | :A man came and asked the moneychanger to give him from the three [kinds of] coins for one zahuv equally, so that the amount of the expensive will be equal to the amount of the inexpensive. | ||
:<math>\scriptstyle\frac{1}{3}X+\frac{1}{4}X+\frac{1}{6}X=1</math> | :<math>\scriptstyle\frac{1}{3}X+\frac{1}{4}X+\frac{1}{6}X=1</math> | ||
| − | |style="text-align:right;"|טו | + | |style="text-align:right;"|טו <big>שאלה</big> יש אצל המחליף ג' מטבעים והזהוב שוה ממטבע אחד ג' דינרי ומן השני ד' ומן השלישי ו‫'<br> |
ובא אדם אחד ובקש למחליף שיתן לו מג' המטבעים בזהוב ויהיה המספר שוה מן היקרים כמו משאינם יקרים{{#annotend:JBea}} | ובא אדם אחד ובקש למחליף שיתן לו מג' המטבעים בזהוב ויהיה המספר שוה מן היקרים כמו משאינם יקרים{{#annotend:JBea}} | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''False Position:'''</span> look for a [number] that has a third, a quarter, and a sixth; it is 12. | |
|style="text-align:right;"|בקש היתרה שיהיה בו שלישית ורבעית וששית והוא י"ב | |style="text-align:right;"|בקש היתרה שיהיה בו שלישית ורבעית וששית והוא י"ב | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\left(\frac{1}{3}\sdot12\right)+\left(\frac{1}{4}\sdot12\right)+\left(\frac{1}{6}\sdot12\right)=9}}</math> | + | :The parts are 9 and this is a dinar. |
| + | :<math>\scriptstyle{\color{blue}{\left(\frac{1}{3}\sdot12\right)+\left(\frac{1}{4}\sdot12\right)+\left(\frac{1}{6}\sdot12\right)=9}}</math> | ||
|style="text-align:right;"|החלקי' הם ט' והוא דינר | |style="text-align:right;"|החלקי' הם ט' והוא דינר | ||
|- | |- | ||
| | | | ||
| − | : | + | :We divide the denominator by it; it is 12-ninths and so he takes from each coin. |
| + | :<math>\scriptstyle{\color{blue}{x=\frac{12}{9}}}</math> | ||
|style="text-align:right;"|נחלק המורה על זה המספר יהיו י"ב תשי' וככה לקח מכל מטבע | |style="text-align:right;"|נחלק המורה על זה המספר יהיו י"ב תשי' וככה לקח מכל מטבע | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{12\sdot\frac{12}{9}=12\sdot\left(1+\frac{1}{3}\right)=12+4=16}}</math> | + | :Another [way]: Say: what is the ratio of 12 to 9; it is the amount plus its third. We add 4 to 12; it is 16. |
| + | :<math>\scriptstyle{\color{blue}{12\sdot\frac{12}{9}=12\sdot\left(1+\frac{1}{3}\right)=12+4=16}}</math> | ||
|style="text-align:right;"|אחר על זאת השאלה שתאמר מה ערך י"ב אל ט' והיה כמות שלישיתו והנה {{#annot:term|178,1206|NQJm}}נוסיף על{{#annotend:NQJm}} י"ב ד' יהיו י"ו | |style="text-align:right;"|אחר על זאת השאלה שתאמר מה ערך י"ב אל ט' והיה כמות שלישיתו והנה {{#annot:term|178,1206|NQJm}}נוסיף על{{#annotend:NQJm}} י"ב ד' יהיו י"ו | ||
|- | |- | ||
| − | | | + | | |
| + | |||
| + | === <span style=color:green>Payment Problem - Digging a Hole</span> === | ||
| + | |||
| | | | ||
|- | |- | ||
| | | | ||
| − | :{{#annot:digging a hole|612|le5j}}16) Reuven hired Shimon to dig for him in the ground 7 in length, 6 in width, 5 in depth, and he will pay him 11 pešiṭim, but he dug 6 in length, 5 in width, 4 in depth. | + | :{{#annot:digging a hole|612|le5j}}16) Question: Reuven hired Shimon to dig for him in the ground 7 in length, 6 in width, 5 in depth, and he will pay him 11 pešiṭim, but he dug 6 in length, 5 in width, 4 in depth. |
:How much should be his payment? | :How much should be his payment? | ||
:<math>\scriptstyle\frac{7\sdot6\sdot5}{11}=\frac{6\sdot5\sdot4}{X}</math> | :<math>\scriptstyle\frac{7\sdot6\sdot5}{11}=\frac{6\sdot5\sdot4}{X}</math> | ||
| − | |style="text-align:right;"|יו | + | |style="text-align:right;"|יו <big>שאלה</big> ראובן שכר שמעון שיחפור לו בקרקע ז' באורך וו' ברוחב וה' בעומק ויתן לו י"א פשיטי‫'<br> |
והוא חפר ו' באורך ה' ברוחב ד' בעומק<br> | והוא חפר ו' באורך ה' ברוחב ד' בעומק<br> | ||
כמה שכרו{{#annotend:le5j}} | כמה שכרו{{#annotend:le5j}} | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\left(7\sdot6\right)\sdot5=42\sdot5=210}}</math> | + | :We do as follows: 7 times 6 is 42. Multiply it by 5, which is the depth; it is 210. |
| + | :<math>\scriptstyle{\color{blue}{\left(7\sdot6\right)\sdot5=42\sdot5=210}}</math> | ||
|style="text-align:right;"|נעשה כך ז' פעמים ו' הם מ"ב כפלם על ה' שהוא העומק ויהיו ר"י | |style="text-align:right;"|נעשה כך ז' פעמים ו' הם מ"ב כפלם על ה' שהוא העומק ויהיו ר"י | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\left(6\sdot5\right)\sdot4=30\sdot4=120}}</math> | + | :We also multiply the second number, which is 6, by 5; it is 30. We multiply it by 4, which is the depth; it is 120. |
| + | :<math>\scriptstyle{\color{blue}{\left(6\sdot5\right)\sdot4=30\sdot4=120}}</math> | ||
|style="text-align:right;"|גם נכפול המספר השני שהוא ו' על ה' והם ל' גם נכפול זה על ד' שהוא העומק יהיו ק"כ | |style="text-align:right;"|גם נכפול המספר השני שהוא ו' על ה' והם ל' גם נכפול זה על ד' שהוא העומק יהיו ק"כ | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> we multiply 11 times 120; it is 1320. Divide it by 210; the result is 6 integers and 60 remains, which is 2-sevenths of one pašuṭ. | |
| − | |style="text-align:right;"|ונכפול י"א פעמי' ק"כ הם אלף וש"כ<br> | + | :<math>\scriptstyle{\color{blue}{x=\frac{11\sdot120}{210}=\frac{1320}{210}=6+\frac{60}{210}=6+\frac{2}{7}}}</math> |
| − | חלקם על ר"י עלו ו' שלמי' ונשאר ס' שהם ב' שבעיות פשוט | + | |style="text-align:right;"|ונכפול י"א פעמי' ‫<ref>89v</ref>ק"כ הם אלף וש"כ<br> |
| + | חלקם על ר"י עלו ו' שלמי' ונשאר ס' שהם ב' שבעיות פשוט וכה"ל | ||
|- | |- | ||
| − | | | + | | |
| + | |||
| + | === <span style=color:green>Buy and Sell Problem</span> === | ||
| + | |||
| | | | ||
|- | |- | ||
| | | | ||
| − | :{{#annot:WP|641|ubS2}}17) A man bought three fifths of a liṭra for one pašuṭ, then he sold four sevenths of a liṭra for one pašuṭ and he earned one pašuṭ. | + | :{{#annot:WP|641|ubS2}}17) Question: A man bought three fifths of a liṭra for one pašuṭ, then he sold four sevenths of a liṭra for one pašuṭ and he earned one pašuṭ. |
:How much money did he have originally?<br> | :How much money did he have originally?<br> | ||
:<math>\scriptstyle\frac{\frac{3}{5}X}{\frac{4}{7}}=X+1</math> | :<math>\scriptstyle\frac{\frac{3}{5}X}{\frac{4}{7}}=X+1</math> | ||
| − | |style="text-align:right;"|יז | + | |style="text-align:right;"|יז <big>שאלה</big> אדם קנה ג' חמשיות ליט' בפשוט ומכר ד' שבעיות ליט' בפשוט והרויח פשוט<br> |
כמה היה ממונו{{#annotend:ubS2}} | כמה היה ממונו{{#annotend:ubS2}} | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''common denominator:'''</span> look for the common denominator: multiply 5 by 7; it is 35. | |
| + | :<math>\scriptstyle{\color{blue}{5\sdot7=35}}</math> | ||
|style="text-align:right;"|בקש המורה הוא ל"ה וכפול ה' על ז‫' | |style="text-align:right;"|בקש המורה הוא ל"ה וכפול ה' על ז‫' | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{x+1=\frac{3}{5}\sdot35=21}}</math> | + | :Its 3-fifths are 21. |
| + | :<math>\scriptstyle{\color{blue}{x+1=\frac{3}{5}\sdot35=21}}</math> | ||
|style="text-align:right;"|והיה ג' חמשיותיו כ"א | |style="text-align:right;"|והיה ג' חמשיותיו כ"א | ||
|- | |- | ||
| | | | ||
| − | : | + | :Its 4-sevenths are 20, so the original amount of money is 20. |
| − | |style="text-align:right;"|וד' שבעיותיו כ' והממון היה כ' וכה" | + | :<math>\scriptstyle{\color{blue}{x=\frac{4}{7}\sdot35=20}}</math> |
| + | |style="text-align:right;"|וד' שבעיותיו כ' והממון היה כ' וכה"ל | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :It seems that he wants to say that 20 pešuṭim were spent. |
| + | |style="text-align:right;"|<sup>נראה שרוצה לו' שהוצ' כ' פשוטי' וזהו</sup> | ||
|- | |- | ||
| − | |||
| | | | ||
| − | + | :{{#annot:WP|641|Tlzb}}18) Question: A man bought four sevenths of a liṭra for one pašuṭ, then he sold five ninth of a liṭra for one pašuṭ and he earned one pašuṭ. | |
| − | |||
| − | :{{#annot:WP|641|Tlzb}}18) A man bought four sevenths of a liṭra for one pašuṭ, then he sold five ninth of a liṭra for one pašuṭ and he earned one pašuṭ. | ||
:How much money did he have originally? | :How much money did he have originally? | ||
:<math>\scriptstyle\frac{\frac{4}{7}X}{\frac{5}{9}}=X+1</math> | :<math>\scriptstyle\frac{\frac{4}{7}X}{\frac{5}{9}}=X+1</math> | ||
| − | |style="text-align:right;"|יח | + | |style="text-align:right;"|יח <big>שאלה</big> אדם קנה ד' שבעיות ליט' בפשוט ומכר ה' תשעיות ליט' בפשוט והרויח פשוט<br> |
כמה היה הממון{{#annotend:Tlzb}} | כמה היה הממון{{#annotend:Tlzb}} | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\frac{4}{7}>\frac{5}{9}}}</math> | + | :It is known that 4-sevenths is greater that 5-ninths. |
| + | :<math>\scriptstyle{\color{blue}{\frac{4}{7}>\frac{5}{9}}}</math> | ||
|style="text-align:right;"|ידוע הוא כי ד' שבעיות אחד הוא יותר מה' תשעיות אחד | |style="text-align:right;"|ידוע הוא כי ד' שבעיות אחד הוא יותר מה' תשעיות אחד | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''common denominator:'''</span> the common denominator is 63. | |
|style="text-align:right;"|והנה המורה ס"ג | |style="text-align:right;"|והנה המורה ס"ג | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{x=\frac{5}{9}\sdot63=35}}</math> | + | :Its 5-ninths are 35. |
| + | :<math>\scriptstyle{\color{blue}{x=\frac{5}{9}\sdot63=35}}</math> | ||
|style="text-align:right;"|וה' תשיעיותיו ל"ה | |style="text-align:right;"|וה' תשיעיותיו ל"ה | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{x+1=\frac{4}{7}\sdot63=36}}</math> | + | :Its 4-sevenths are 36. |
| + | :<math>\scriptstyle{\color{blue}{x+1=\frac{4}{7}\sdot63=36}}</math> | ||
|style="text-align:right;"|וד' שבעיותיו ל"ו | |style="text-align:right;"|וד' שבעיותיו ל"ו | ||
|- | |- | ||
| | | | ||
| − | :Check: < | + | :<span style=color:green>'''Check:'''</span> you can check this: as he buys 4-sevenths of a liṭra for one pašuṭ, and his money is 35, he has 20 liṭra. |
| − | |style="text-align:right;"|ותוכל לבחון זה כי אחר שקנה ד' שבעיות ליט' בפשוט ממונו ל"ה הנה יש לו כ' ליט‫' | + | |style="text-align:right;"|ותוכל לבחון זה כי אחר שקנה ד' שבעיות ליט' בפשוט ממונו ל"ה הנה יש לו כ' ליט‫' |
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | *If he earned | + | :Convert them into ninths; they are 180. |
| + | |style="text-align:right;"|עשה מהם תשעיו' יהיו ק"פ | ||
| + | |- | ||
| + | | | ||
| + | :Divide this number by 5, because he sold 5-ninths for one pašuṭ; you receive 36. | ||
| + | :<math>\scriptstyle{\color{blue}{\frac{\frac{4}{7}\sdot35}{\frac{5}{9}}=\frac{20}{\frac{5}{9}}=\frac{20\sdot9}{5}=\frac{180}{5}=36=35+1}}</math> | ||
| + | |style="text-align:right;"|חלק זה המספר על ה' כי ה' תשעיו' מכר בפשוט יעלו בידך ל"ו | ||
| + | |- | ||
| + | | | ||
| + | *If it is said that he earned 2 pešiṭim: | ||
:<math>\scriptstyle\frac{\frac{4}{7}X}{\frac{5}{9}}=X+2</math> | :<math>\scriptstyle\frac{\frac{4}{7}X}{\frac{5}{9}}=X+2</math> | ||
|style="text-align:right;"|ואילו אמר כי הרויח ב"פ | |style="text-align:right;"|ואילו אמר כי הרויח ב"פ | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{x=2\sdot35}}</math> | + | :Multiply them by 35 and they are the pešuṭim he spent. |
| − | |style="text-align:right;"|כפלם על ל"ה יהיו הפשוטי' שהוציא | + | :<math>\scriptstyle{\color{blue}{x=2\sdot35}}</math> |
| + | |style="text-align:right;"|כפלם על ל"ה יהיו <sup>הפשוטי' שהוציא</sup> | ||
|- | |- | ||
| | | | ||
| − | + | :<math>\scriptstyle{\color{blue}{\frac{\frac{4}{7}\sdot\left(2\sdot35\right)}{\frac{5}{9}}=\frac{40}{\frac{5}{9}}=\left(2\sdot35\right)+2}}</math> | |
| − | |style="text-align:right;"|כי | + | |style="text-align:right;"|<sup>כי הממון יהיה מ' ליט‫'</sup> |
|- | |- | ||
| | | | ||
| − | *If he earned | + | *If it is said [that he earned] 3 pešiṭim: |
:<math>\scriptstyle\frac{\frac{4}{7}X}{\frac{5}{9}}=X+3</math> | :<math>\scriptstyle\frac{\frac{4}{7}X}{\frac{5}{9}}=X+3</math> | ||
|style="text-align:right;"|ואם אמר ג"פ | |style="text-align:right;"|ואם אמר ג"פ | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{x=3\sdot35}}</math> | + | :He multiplies them by 35. |
| − | |style="text-align:right;"|יכפלם על ל"ה | + | :<math>\scriptstyle{\color{blue}{x=3\sdot35}}</math> |
| + | |style="text-align:right;"|יכפלם על ל"ה | ||
|- | |- | ||
| − | | | + | | |
| − | + | :And so on for any number. | |
| + | |style="text-align:right;"|וככה עד סוף החשבון | ||
|- | |- | ||
| | | | ||
| − | == Exchange Problems == | + | == <span style=color:green>Exchange Problems</span> == |
| | | | ||
|- | |- | ||
| | | | ||
| − | :19) <span style=color: | + | :19) <span style=color:green>'''The Rule of Three:'''</span> The rule of all calculations is in three categories: geometry; weights; arithmetic. |
| − | + | |style="text-align:right;"|יט <big>כלל</big> כל החשבונות הוא על שלשה פנים במדה או במשקל או {{#annot:term|365,1174|Pv46}}במספר{{#annotend:Pv46}} | |
| − | |style="text-align:right;"|יט | + | |- |
| − | על כן נאמר | + | | |
| − | אם ישאל לך שום חשבון שיהיה בו אחד מאלו הג' מינים<br> | + | :Therefore, we specify the appropriate rules for all three. |
| − | נרבה אותו הדבר שנרצה | + | |style="text-align:right;"|על כן נאמר הריגולי' הנאותים לשלשתם |
| + | |- | ||
| + | | | ||
| + | :If one asks you for a calculation in one of the three categories: we multiply the thing we want to know by the one that is not of the same species, then divide it by the third. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{a_1:a_2=a_3:x\longrightarrow x=\frac{a_2\sdot a_3}{a_1}}}</math> | ||
| + | |style="text-align:right;"|אם ישאל לך שום חשבון שיהיה בו אחד מאלו הג' מינים<br> | ||
| + | נרבה אותו הדבר שנרצה לידע כנגד אותה שאיננה ממינה עצמה ממש<br> | ||
ותחלקנה על השלשי‫' | ותחלקנה על השלשי‫' | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:two currencies|632|CUc1}}If 7 of Pisa are worth 9 of Cortona, how many of Cortona are 100 liṭra of Pisa worth? | + | *{{#annot:two currencies|632|CUc1}}Example: If 7 of Pisa are worth 9 of Cortona, how many of Cortona are 100 liṭra of Pisa worth? |
:<math>\scriptstyle\frac{7}{9}=\frac{100}{X}</math> | :<math>\scriptstyle\frac{7}{9}=\frac{100}{X}</math> | ||
|style="text-align:right;"|המשל בזה אם ז' פיסט' שוים ט' קורטוניס כמה ישוו ק' ליט' מפיסני' לקורטו‫'{{#annotend:CUc1}} | |style="text-align:right;"|המשל בזה אם ז' פיסט' שוים ט' קורטוניס כמה ישוו ק' ליט' מפיסני' לקורטו‫'{{#annotend:CUc1}} | ||
|- | |- | ||
| | | | ||
| − | : | + | :You already know that the thing of Cortona is not of the same species, so we multiply 9 times 100; it is 900. |
| − | |style="text-align:right;"|כבר ידעת כי הקורטו' הוא הדבר שאינה ממינה עצמה ממש לכן נרבה ט' פעמי' ק' יהיו תת"ק | + | |style="text-align:right;"|כבר ידעת כי הקורטו' הוא הדבר שאינה ממינה עצמה ממש לכן נרבה ט' פעמי' ק' יהיו תת"ק |
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :Divide it by 7; it is 128 liṭra and 11 dinar and this is the value of 100 liṭra of Pisa in Cortona. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{x=\frac{9\sdot100}{7}=\frac{900}{7}=128+\frac{4}{7}=128+\frac{11}{20}+\frac{3}{7\sdot20}}}</math> |
| + | |style="text-align:right;"|וחלקם על ז' קכ"ח ליט' וי"א דינרי' והם וכך ישוו הק' ליט' מפיסני' לקורטו‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | :Know that if 7 of Pisa equal 9 of Cortona: 7 dinar of Pisa are equal to 9 dinar of Cortona; 7 liṭra of Pisa are equal 9 liṭra of Cortona; 700 liṭra of Pisa are equal to 900 liṭra of Cortona. |
| − | |style="text-align:right;"|הז' דינרי' פיסני' שוים ט' דינרי' קורטוני‫' | + | |style="text-align:right;"|ודע כי אם ז' פיסני' שוים ט' קורטו' הז' דינרי' פיסני' שוים ט' דינרי' קורטוני' והז' ליט' מפיסני' שוים ט' ליט' קורטו' ות"ש ליט' מפיסני' שוים תת"ק ליט' קורטו‫' |
|- | |- | ||
| − | | | + | |? |
| − | + | |style="text-align:right;"|וכן יבא בין פפריני' ופריביסיני' ולכל [חשבון] כזה | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | ::: | + | :20) <span style=color:green>'''Rule of Three - with fractions:'''</span> If you are asked for a calculation of these three categories, where both parts involve fractions: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כ <big>אם</big> ישאל לך חשבון שיהיה בו מאלו הג' מינים ולפני הב' חלקי' יהיו שבורים |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :We know what number has these fractions. |
| + | |style="text-align:right;"|נדע באיזה המספר ימצאו אלו השבורים | ||
|- | |- | ||
| | | | ||
| − | : | + | :Once you find the number in which these fractions are found, we multiply both parts by that number, in which these fractions are found. |
| − | + | |style="text-align:right;"|ואחר שידעת באי זה המספר ימצאו אלו השבורים נרבה הב' חלקי' באותו המספר שימצאו בו אלו השבורים | |
| − | |style="text-align:right;"| | ||
| − | |||
| − | ואחר שידעת באי זה המספר ימצאו אלו השבורים נרבה הב' חלקי' באותו המספר שימצאו בו אלו השבורים | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :Then, we multiply the thing we want to know by the thing that is not of its own kind and divide it by the third. |
| − | + | |style="text-align:right;"|אחרי כן נרבה אותו הדבר שנרצה לדעת כנגד אותו הדבר שאנינה ממינה עצמה ונחלק אותה על השלישית וכה"ל | |
| − | |style="text-align:right;"| | ||
| − | |||
| − | אחרי כן נרבה אותו הדבר שנרצה | ||
| − | ונחלק אותה על השלישית | ||
|- | |- | ||
| − | | | + | | colspan="2"| |
| + | :<math>\scriptstyle{\color{OliveGreen}{\frac{a_1}{b_1}:\frac{a_2}{b_2}=a_3:x\longrightarrow\left[\frac{a_1}{b_1}\sdot\left(b_1\sdot b_2\right)\right]:\left[\frac{a_2}{b_2}\sdot\left(b_1\sdot b_2\right)\right]=a_3:x\longrightarrow x=\frac{\left[\frac{a_2}{b_2}\sdot\left(b_1\sdot b_2\right)\right]\sdot a_3}{\frac{a_1}{b_1}\sdot\left(b_1\sdot b_2\right)}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :21) <span style=color:green>'''Rule of Three - with one fraction:'''</span> If you are asked for another calculation in which one part involves fractions: | ||
| + | |style="text-align:right;"|כא <big>ואם</big> נשאל לך חשבון אחר שיהיה שבור מחלק אחד | ||
| + | |- | ||
| + | | | ||
| + | :We multiply both parts by that number, i.e. by the [denominator of] the fraction. | ||
| + | |style="text-align:right;"|נרבה הב' חלקי' באותו המספר דהיינו עם אותו השבור | ||
| + | |- | ||
| + | | | ||
| + | :Then, we multiply the thing we want to know by the thing that is not of its kind and divide it by the third. | ||
| + | |style="text-align:right;"|אחרי כן נרבה אותו הדבר שנרצה לידע כנגד אותו הדבר שאנינה ממינה ונחלק אותה על השלישית | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\frac{a_1}{b_1}:a_2=a_3:x \longrightarrow\left(\frac{a_1}{b_1}\sdot b_1\right):\left(a_2\sdot b_1\right)=a_3:x\longrightarrow x=\frac{\left(a_2\sdot b_1\right)\sdot a_3}{\frac{a_1}{b_1}\sdot b_1}}}</math> | ||
| + | |- | ||
| + | |We give an example for the two cases - one for a calculation in which one part involves a fraction and the other in which the two parts involve fractions: | ||
|style="text-align:right;"|ונשים המשל בשני פנים האחד בעבור החשבון שהוא שבור מחלק אחד והאחר שהוא שבור מב' חלקי‫' | |style="text-align:right;"|ונשים המשל בשני פנים האחד בעבור החשבון שהוא שבור מחלק אחד והאחר שהוא שבור מב' חלקי‫' | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:two currencies|632|ppYb}}If | + | *{{#annot:two currencies|632|ppYb}}If 5 and a quarter of Pisa are worth 7 of Cortona, how many of Cortona are 1000 liṭra of Pisa worth? |
:<math>\scriptstyle\frac{5+\frac{1}{4}}{7}=\frac{1000}{X}</math> | :<math>\scriptstyle\frac{5+\frac{1}{4}}{7}=\frac{1000}{X}</math> | ||
| − | |style="text-align:right;"|אם ה' פיסני' ורביע שוים ז' | + | |style="text-align:right;"|אם ה' פיסני' ורביע שוים ז' קורטונו' כמה ישוו אלף ליט' מפיסני‫'{{#annotend:ppYb}} |
|- | |- | ||
| | | | ||
| − | : | + | :This is a calculation in which one part involves a fraction and this fraction is a quarter. So, we multiply both parts by 4. |
|style="text-align:right;"|וזה החשבון הוא שבור מחלק אחד וזה השיבור הוא הרביע<br> | |style="text-align:right;"|וזה החשבון הוא שבור מחלק אחד וזה השיבור הוא הרביע<br> | ||
לכן נרבה הב' חלקי' עם ד‫' | לכן נרבה הב' חלקי' עם ד‫' | ||
|- | |- | ||
| | | | ||
| − | :::<math>\scriptstyle{\color{blue}{4\sdot\left(5+\frac{1}{4}\right)=21}}</math> | + | :Say: 4 times 5 pisani and a quarter; the result is 21 pisani. |
| + | :<math>\scriptstyle{\color{blue}{4\sdot\left(5+\frac{1}{4}\right)=21}}</math> | ||
|style="text-align:right;"|ואמור ד' פעמי' ה' פיסני' ורביע יבוא כ"א פיסני‫' | |style="text-align:right;"|ואמור ד' פעמי' ה' פיסני' ורביע יבוא כ"א פיסני‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | :4 times 7 corto; the result is 28 corto. |
| + | :<math>\scriptstyle{\color{blue}{4\sdot7=28}}</math> | ||
|style="text-align:right;"|וד' פעמי' ז' קורטו' בא כ"ח קורטו‫' | |style="text-align:right;"|וד' פעמי' ז' קורטו' בא כ"ח קורטו‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | :So, 21 pisani are worth 28 of corto. |
|style="text-align:right;"|הרי שכ"א פיסני' שוים כ"ח קורטו‫' | |style="text-align:right;"|הרי שכ"א פיסני' שוים כ"ח קורטו‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | :We reduce by 7 [lit. divide by a seventh], because both parts has a seventh. |
| − | |style="text-align:right;"|ונחלק לשביע בעבור שבשני החלקי' ימצא שביע | + | |style="text-align:right;"|ונחלק לשביע בעבור ‫<ref>90r</ref>שבשני החלקי' ימצא שביע |
|- | |- | ||
| | | | ||
| − | : | + | :The seventh of 21 is 3. |
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{7}\sdot21=3}}</math> | ||
|style="text-align:right;"|השביע מכ"א הוא ג‫' | |style="text-align:right;"|השביע מכ"א הוא ג‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | :The seventh of 28 is 4. |
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{7}\sdot28=4}}</math> | ||
|style="text-align:right;"|השביע מכ"ח הוא ד‫' | |style="text-align:right;"|השביע מכ"ח הוא ד‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | :We find that 3 pisani are worth 4 corto. |
|style="text-align:right;"|נמצא שג' פיסני' שוים ד' קורטו‫' | |style="text-align:right;"|נמצא שג' פיסני' שוים ד' קורטו‫' | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> We want to know how much are a thousand liṭra of Pisa worth. The pisani is the thing we want to know and the corto is the thing that is not of its own type. | |
| − | |style="text-align:right;"|ואנחנו נרצה לידע כמה ישוו אלף ליט' מפיסני' הפיסני הוא הדבר שנרצה לידע והקורטו' הוא הדבר שאנינה ממינה עצמה | + | :<math>\scriptstyle{\color{blue}{3:4=1000:X}}</math> |
| + | |style="text-align:right;"|ואנחנו נרצה לידע כמה ישוו אלף ליט' מפיסני‫'<br> | ||
| + | הפיסני הוא הדבר שנרצה לידע והקורטו' הוא הדבר שאנינה ממינה עצמה | ||
|- | |- | ||
| | | | ||
| − | : | + | :Therefore, we multiply 4 times a thousand; the result is 4 thousand of Cortona. |
| − | + | |style="text-align:right;"|לכן נרבה ד' פעמים אלף יבא ד' אלפי' מקורטו‫' | |
| − | |style="text-align:right;"|לכן נרבה ד' פעמים אלף יבא ד' אלפי' מקורטו | ||
| − | |||
|- | |- | ||
| | | | ||
| − | *{{#annot:two measures|867|p0EU}}If | + | :We divide it by 3; the result is 1333 liṭra, 6 dinar and 8 pešuṭim and so the thousand liṭra of of Pisa are worth in Cortona. |
| + | :<math>\scriptstyle{\color{blue}{X=\frac{1000\sdot4}{3}=\frac{4000}{3}=1333+\frac{6}{20}+\frac{\frac{8}{12}}{20}}}</math> | ||
| + | |style="text-align:right;"|ונחלק בג' שיבא אלף ושל"ג ליט' וו' דינרי' וח"פ וכן ישוו אלף ליט' מפיסני לקורטוט‫' | ||
| + | |- | ||
| + | |We give an example of a calculation in which both parts involve fractions: | ||
| + | |style="text-align:right;"|ונשים המשל אל החשבון שהוא שבור מב' חלקיו | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:two measures|867|p0EU}}If 7 silver ounces and a third are worth 73 liṭra and 5 dinar of Pisa, how many are 19 silver ounces worth? | ||
:<math>\scriptstyle\frac{7+\frac{1}{3}}{73+\frac{1}{4}}=\frac{19}{X}</math> | :<math>\scriptstyle\frac{7+\frac{1}{3}}{73+\frac{1}{4}}=\frac{19}{X}</math> | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אם ז' אונקיו' ושליש כסף שוים ע"ג ליט' וה' דינרי' מפיסני‫'<br> |
כמה ישוו הי"ט אונקיו' כסף לפי זה החשבון{{#annotend:p0EU}} | כמה ישוו הי"ט אונקיו' כסף לפי זה החשבון{{#annotend:p0EU}} | ||
|- | |- | ||
| | | | ||
| − | : | + | :These fractions are a third and a quarter and they are found in 12. |
| − | |style="text-align:right;"|ואלו השבורים הם שליש ורביע אנה ימצאו | + | |style="text-align:right;"|ואלו השבורים הם שליש ורביע אנה ימצאו בי"ב |
| − | |||
|- | |- | ||
| | | | ||
| − | :::<math>\scriptstyle{\color{blue}{12\sdot\left(7+\frac{1}{3}\right)=88}}</math> | + | :So, we must multiply both parts by 12. |
| + | |style="text-align:right;"|לכן יש לנו לרבות הב' חלקי' בי"ב | ||
| + | |- | ||
| + | | | ||
| + | :Say: 12 times 7 ounces and a third; the result is 88 silver ounces. | ||
| + | :<math>\scriptstyle{\color{blue}{12\sdot\left(7+\frac{1}{3}\right)=88}}</math> | ||
|style="text-align:right;"|ואמור י"ב פעמי' ז' אונקיו' ושליש יבא פ"ח אונקיו' כסף | |style="text-align:right;"|ואמור י"ב פעמי' ז' אונקיו' ושליש יבא פ"ח אונקיו' כסף | ||
|- | |- | ||
| | | | ||
| − | : | + | :12 times 73 liṭra and 5 dinar are 879 liṭra of Pisa. |
| + | :<math>\scriptstyle{\color{blue}{12\sdot\left(73+\frac{1}{4}\right)=879}}</math> | ||
|style="text-align:right;"|וי"ב פעמי' ע"ג ליט' וה' דינרי' יהיו תתע"ט ליט' מפסני‫' | |style="text-align:right;"|וי"ב פעמי' ע"ג ליט' וה' דינרי' יהיו תתע"ט ליט' מפסני‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | :Therefore, 88 silver ounces are worth 879 liṭra. |
|style="text-align:right;"|הרי שפ"ח אונקיות כסף שוים תתע"ט ליט‫' | |style="text-align:right;"|הרי שפ"ח אונקיות כסף שוים תתע"ט ליט‫' | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> We want to know how much are 19 silver ounces worth. | |
| + | :<math>\scriptstyle{\color{blue}{88:879=19:X}}</math> | ||
|style="text-align:right;"|ונרצה לידע כמה ישוו הי"ט אונקיו' כסף | |style="text-align:right;"|ונרצה לידע כמה ישוו הי"ט אונקיו' כסף | ||
|- | |- | ||
| | | | ||
| − | : | + | :We multiply 19 times 879 liṭra; they are 16701. |
| − | + | |style="text-align:right;"|נרבה י"ט פעמי' תתע"ט ליט' יהיו י"ו אלפי' ותש"א | |
| − | |style="text-align:right;"|נרבה י"ט פעמי' תתע"ט ליט' יהיו י"ו אלפי' ותש"א | ||
| − | |||
|- | |- | ||
| − | |} | + | | |
| − | + | :Divide them by 88; the result is 189 liṭra, 15 dinar, 8 pešuṭim and 2 parts of 11 and so the 19 silver ounce are worth. | |
| + | :<math>\scriptstyle{\color{blue}{X=\frac{19\sdot879}{88}=\frac{16701}{88}=\frac{16701}{8\sdot11}=189+\frac{15}{20}+\frac{\frac{8+\frac{2}{11}}{12}}{20}}}</math> | ||
| + | |style="text-align:right;"|חלקם בפ"ח יבא קפ"ט ליט' וט"ו די' וח"פ וב' חלקי' מי"א וכן ישוו הי"ט אונקיו' כסף באותו החשבון | ||
|- | |- | ||
| | | | ||
| − | == Interest and Pricing Problems == | + | == <span style=color:green>Interest and Pricing Problems</span> == |
| | | | ||
|- | |- | ||
| − | |'''Find the Time''' | + | |<span style=color:green>'''Find the Time'''</span> |
| | | | ||
|- | |- | ||
| | | | ||
| − | :{{#annot:for some liṭra|660|XXox}}22) | + | :{{#annot:for some liṭra|660|XXox}}22) Question: one kikkar yields 36 liṭra and 18 dinar a year. |
:How long will it take 65 liṭra to yield the same? | :How long will it take 65 liṭra to yield the same? | ||
:<math>\scriptstyle\frac{\left(36\sdot20\right)+18}{12\sdot100}=\frac{\left(36\sdot20\right)+18}{X\sdot65}</math> | :<math>\scriptstyle\frac{\left(36\sdot20\right)+18}{12\sdot100}=\frac{\left(36\sdot20\right)+18}{X\sdot65}</math> | ||
| − | |style="text-align:right;"|כב | + | |style="text-align:right;"|כב <big>שאלה</big> הככר ירויח השנה ל"ו ליט' וי"ח די' נוסף<br> |
| − | הס"ה ליט' בכמה זמן ירויחו | + | הס"ה ליט' בכמה זמן ירויחו כהם{{#annotend:XXox}} |
| + | |- | ||
| + | | | ||
| + | :<span style=color:green>'''Rule of Three:'''</span> multiply the 100 liṭra by the 12 months of the year, say: 12 times 100 are 1200. | ||
| + | |style="text-align:right;"|תרבה הק' ליט' כנגד י"ב חדשי השנה ואמור י"ב פעמי' ק' חדשים הם אלף ור‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | :Divide them by 65; each part receives 18 months, 13 days and 11 parts of 13 parts of a day. |
| − | + | :<math>\scriptstyle{\color{blue}{x=\frac{12\sdot100}{65}=\frac{1200}{65}=18+\frac{13}{30}+\frac{\frac{11}{13}}{30}}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|חלקם על ס"ה יבא לכל חלק י"ח חדשי' וי"ג ימים וי"א חלקי' מי"ג חלקי' ביום |
| − | חלקם על ס"ה יבא לכל חלק י"ח חדשי' וי"ג ימים וי"א חלקי' מי"ג חלקי' ביום | ||
| − | |||
|- | |- | ||
| − | |||
| | | | ||
| + | :We find that 65 liṭra yield in 18 months, 13 days, and 11 parts of 13 of a day the same as one kikkar yields in one year. | ||
| + | |style="text-align:right;"|נמצא שבי"ח חדשי' וי"ג ימי' וי"א חלקי' מי"ג ביום ירויחו ס"ה ליט' כלכך כמו שירויח הככר השנה | ||
|- | |- | ||
| + | |<span style=color:green>'''Find the Earned Interest'''</span> | ||
| | | | ||
| − | :{{#annot:for some liṭra, some months|638|uzR3}}23) I lent | + | |- |
| − | :How | + | | |
| + | :{{#annot:for some liṭra, some months|638|uzR3}}23) If a man says: I lent some money at 3 pešuṭim a month for one liṭra. | ||
| + | :How much will 60 liṭra make in 8 months? | ||
:<math>\scriptstyle\frac{3}{1\sdot1}=\frac{\left(20\sdot12\right)\sdot X}{8\sdot60}</math> | :<math>\scriptstyle\frac{3}{1\sdot1}=\frac{\left(20\sdot12\right)\sdot X}{8\sdot60}</math> | ||
| − | |style="text-align:right;"|כג | + | |style="text-align:right;"|כג <big>שאלה</big> אם יאמר אדם הלותי מעות לחשבון גפה"ח <sup>הליט' החדש</sup><br> |
כמה יבואו הס' ליט' ח' חדשים{{#annotend:uzR3}} | כמה יבואו הס' ליט' ח' חדשים{{#annotend:uzR3}} | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{3\sdot8=24}}</math> | + | :Multiply the interest by the months and say: 3 times 8 are 24 [pešuṭim], which are 2 dinar. |
| + | :<math>\scriptstyle{\color{blue}{3\sdot8=24}}</math> | ||
|style="text-align:right;"|תרבה מעות הרבית עם החדשים ואמור ג' פעמי' ח' כ"ד שהם ב' דינרי‫' | |style="text-align:right;"|תרבה מעות הרבית עם החדשים ואמור ג' פעמי' ח' כ"ד שהם ב' דינרי‫' | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{2\sdot60=120 | + | :Then, multiply these 2 dinar by 60 liṭra and say: 60 times 2 dinar are 120 dinar, which are 6 liṭra. |
| − | + | :<math>\scriptstyle{\color{blue}{2\sdot60=120=20\sdot6}}</math> | |
| − | |style="text-align:right;"|אחר כן תרבה אלו הב' די' עם הס' ליט' ואמור ס' פעמי' ב' דינרי' הם ק"כ די' שהם ו' ליט‫'< | + | |style="text-align:right;"|אחר כן תרבה אלו הב' די' עם הס' ליט' ואמור ס' פעמי' ב' דינרי' הם ק"כ די' שהם ו' ליט‫' |
| − | נמצא שבס' ליט' לחשבון גפה"ח יבוא ו' ליט' לח' חדשים וכה | + | |- |
| + | | | ||
| + | :We find that 60 liṭra at 3 pešuṭim for one liṭra a month will make 6 liṭra in 8 months. | ||
| + | :<math>\scriptstyle{\color{blue}{X=6}}</math> | ||
| + | |style="text-align:right;"|נמצא שבס' ליט' לחשבון גפה"ח יבוא ו' ליט' לח' חדשים וכה"ל | ||
|- | |- | ||
| − | |'''Find the Fund''' | + | |<span style=color:green>'''Find the Fund'''</span> |
| | | | ||
|- | |- | ||
| | | | ||
| − | :{{#annot:liṭra for one day|666|Habg}}24) I lent one liṭra for so and so | + | :{{#annot:liṭra for one day|666|Habg}}24) If a man asks you: I lent some money at one liṭra for so and so a month. |
:How many liṭra will yield one pašuṭ a day? | :How many liṭra will yield one pašuṭ a day? | ||
:<math>\scriptstyle\frac{a}{30\sdot1}=\frac{1}{1\sdot X}</math> | :<math>\scriptstyle\frac{a}{30\sdot1}=\frac{1}{1\sdot X}</math> | ||
| − | |style="text-align:right;"|כד | + | |style="text-align:right;"|כד <big>אם</big> ישאלך אדם הלותי מעות <sup>הליט'</sup> לחשבון כך וכך <sup>החדש</sup><br> |
| − | כמה ליט' ירויחו היום | + | כמה ליט' ירויחו היום א"פ{{#annotend:Habg}} |
|- | |- | ||
| | | | ||
| − | : | + | :We divide 30 liṭra by the amount of money that one liṭra makes a month. |
| − | + | :<math>\scriptstyle{\color{blue}{X=\frac{30}{a}}}</math> | |
|style="text-align:right;"|נחלק ל' ליט' בכל כך חלקי' כמו המעות שירויח הליט' החדש | |style="text-align:right;"|נחלק ל' ליט' בכל כך חלקי' כמו המעות שירויח הליט' החדש | ||
|- | |- | ||
| | | | ||
| − | + | *{{#annot:liṭra for one day|666|xRwx}}Example: I lent one liṭra for 2 pešuṭim and a half a month. | |
| − | : | + | :You want to know how many liṭra will yield one pašuṭ a day? |
| − | + | :<math>\scriptstyle\frac{2+\frac{1}{2}}{30\sdot1}=\frac{1}{1\sdot X}</math> | |
|style="text-align:right;"|המשל בזה הרי שהלותי הליט' בב' פשוטי' וחצי החדש<br> | |style="text-align:right;"|המשל בזה הרי שהלותי הליט' בב' פשוטי' וחצי החדש<br> | ||
| − | ותרצה לידע כמה ליט' ירויחו היום | + | ותרצה לידע כמה ליט' ירויחו היום א"פ{{#annotend:xRwx}} |
| + | |- | ||
| + | | | ||
| + | :We divide 30 liṭra by 2 and half; the result is 12 liṭra. | ||
| + | :<math>\scriptstyle{\color{blue}{X=\frac{30}{2+\frac{1}{2}}=12}}</math> | ||
| + | |style="text-align:right;"|נחלק ל' ליט' בב' וחצי שיבוא י"ב ליט‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | :We find that 12 liṭra will make one pešuṭim a day. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|נמצא שבי"ב ליט' ירויחו היום א"פ וכה"ל |
| − | נמצא שבי"ב ליט' ירויחו היום | ||
|- | |- | ||
| − | |'''Find the Time''' | + | |<span style=color:green>'''Find the Time'''</span> |
| | | | ||
|- | |- | ||
| | | | ||
| − | :{{#annot:days, for one liṭra|660|1jH8}}25) I lent one liṭra for so and so a month. | + | :{{#annot:days, for one liṭra|660|1jH8}}25) If a man asks you: I lent one liṭra for so and so a month. |
| − | :In how many days will one liṭra yield one? | + | :In how many days will one liṭra yield one pašuṭ? |
:<math>\scriptstyle\frac{a}{30\sdot1}=\frac{1}{X\sdot1}</math> | :<math>\scriptstyle\frac{a}{30\sdot1}=\frac{1}{X\sdot1}</math> | ||
| − | |style="text-align:right;"|כה | + | |style="text-align:right;"|כה <big>אם</big> ישאלך אדם הלותי הליט' לחשבון כך וכך החודש בכמה ימים ירויח הליט' א"פ{{#annotend:1jH8}} |
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle X=\frac{30}{a}</math> | + | :Divide 30 days by the amount of money that one liṭra yields a month. |
| + | :<math>\scriptstyle X=\frac{30}{a}</math> | ||
|style="text-align:right;"|חלק ל' יום בכל כך חלקי' כמו המעות שירויח הליט' החדש | |style="text-align:right;"|חלק ל' יום בכל כך חלקי' כמו המעות שירויח הליט' החדש | ||
|- | |- | ||
| | | | ||
| − | + | *{{#annot:days, for one liṭra|660|xUoL}}Example: you lent [one liṭra] for 3 [a month]. | |
| − | : | + | :You want to know in how many days will the liṭra yield one pašuṭ? |
| − | + | :<math>\scriptstyle\frac{3}{30\sdot1}=\frac{1}{X\sdot1}</math> | |
| − | |style="text-align:right;"|המשל בזה הרי שהלוית לחשבון ג' ותרצה לידע כמה ימים ירויח הליט' | + | |style="text-align:right;"|המשל בזה הרי שהלוית לחשבון ג' ותרצה לידע כמה ימים ירויח הליט' א"פ{{#annotend:xUoL}} |
|- | |- | ||
| | | | ||
| − | : | + | :Divide 30 days into 3 parts; the result is 10 days. |
| − | |style="text-align:right;"|חלק ל' יום בג' חלקי' שיבוא י' ימים | + | :<math>\scriptstyle{\color{blue}{x=\frac{30}{3}=10}}</math> |
| + | |style="text-align:right;"|חלק ל' יום בג' חלקי' שיבוא י' ימים | ||
|- | |- | ||
| − | |||
| | | | ||
| + | :So, the liṭra will yield one pašuṭ in 10 days. | ||
| + | |style="text-align:right;"|הרי שבי' ימים ירויח הליט' א"פ | ||
|- | |- | ||
| + | |<span style=color:green>'''Find the Fund'''</span> | ||
| | | | ||
| − | :{{#annot:liṭra for one day|666|pclr}}26) | + | |- |
| + | | | ||
| + | :{{#annot:liṭra for one day|666|pclr}}26) If a man asks you: one kikkar yields so and so liṭra a year. | ||
:How many liṭra will yield one pašuṭ a day? | :How many liṭra will yield one pašuṭ a day? | ||
:<math>\scriptstyle\frac{\left(20\sdot12\right)\sdot a}{360\sdot100}=\frac{1}{1\sdot X}</math> | :<math>\scriptstyle\frac{\left(20\sdot12\right)\sdot a}{360\sdot100}=\frac{1}{1\sdot X}</math> | ||
| − | |style="text-align:right;"|כו | + | |style="text-align:right;"|כו <big>אם</big> ישאלך אדם הככר ירויח כל השנה כל כך ליט‫'<br> |
| − | כמה ליט' ירויחו היום | + | כמה ליט' ירויחו היום א"פ{{#annotend:pclr}} |
|- | |- | ||
| | | | ||
| − | : | + | :Divide 150 liṭra by the number of liṭra that one kikkar yields a year. |
| − | + | :<math>\scriptstyle{\color{blue}{X=\frac{150}{a}}}</math> | |
|style="text-align:right;"|חלק ק"נ ליט' בכל כך חלקי' כמו הליט' שירויח הככר השנה | |style="text-align:right;"|חלק ק"נ ליט' בכל כך חלקי' כמו הליט' שירויח הככר השנה | ||
|- | |- | ||
| | | | ||
| − | + | *{{#annot:liṭra for one day|666|Vv31}}Example: one kikkar yields 12 liṭra a year. | |
| − | : | + | :You want to know how many liṭra will yield one pašuṭ a day? |
| − | + | :<math>\scriptstyle\frac{\left(20\sdot12\right)\sdot12}{360\sdot100}=\frac{1}{1\sdot X}</math> | |
|style="text-align:right;"|המשל בזה הככר ירויח י"ב ליט' השנה<br> | |style="text-align:right;"|המשל בזה הככר ירויח י"ב ליט' השנה<br> | ||
| − | ותרצה לידע כמה ליט' ירויחו היום | + | ותרצה לידע כמה ליט' ירויחו היום א"פ{{#annotend:Vv31}} |
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{X=\frac{150}{12}=12+\frac{1}{2}}}</math> | + | :Divide 150 liṭra into 12 parts; the result is 12 and a half. |
| − | |style="text-align:right;"|חלק ק"נ ליט' בי"ב חלקי' שיבוא | + | :<math>\scriptstyle{\color{blue}{X=\frac{150}{12}=12+\frac{1}{2}}}</math> |
| − | + | |style="text-align:right;"|חלק ק"נ ליט' בי"ב חלקי' שיבוא ‫<ref>90v</ref>י"ב וחצי | |
|- | |- | ||
| − | |||
| | | | ||
| + | :So, 12 liṭra and a half will yield one pašuṭ a day. | ||
| + | |style="text-align:right;"|הרי שבי"ב ליט' וחצי ירויחו ביום א"פ וכה"ל | ||
|- | |- | ||
| + | |<span style=color:green>'''Find the Time'''</span> | ||
| | | | ||
| − | :{{#annot:days, for one liṭra|660|JrEQ}}27) | + | |- |
| + | | | ||
| + | :{{#annot:days, for one liṭra|660|JrEQ}}27) If a man asks you: one kikkar yields so and so liṭra a year. | ||
:In how many days will one liṭra yield one pašuṭ? | :In how many days will one liṭra yield one pašuṭ? | ||
:<math>\scriptstyle\frac{\left(20\sdot12\right)\sdot a}{360\sdot100}=\frac{1}{X\sdot1}</math> | :<math>\scriptstyle\frac{\left(20\sdot12\right)\sdot a}{360\sdot100}=\frac{1}{X\sdot1}</math> | ||
| − | |style="text-align:right;"|כז | + | |style="text-align:right;"|כז <big>אם</big> ישאלך אדם הככר ירויח כל כך ליט' השנה<br> |
| − | בכמה ימים ירויח הליט' | + | בכמה ימים ירויח הליט' א"פ{{#annotend:JrEQ}} |
|- | |- | ||
| | | | ||
| − | : | + | :Divide 150 days by the number of liṭra that the kikkar yields a year. |
| − | + | :<math>\scriptstyle{\color{blue}{X=\frac{150}{a}}}</math> | |
|style="text-align:right;"|חלק ק"נ ימים בכל כך חלקי' כמו הליט' שירויח הככר השנה | |style="text-align:right;"|חלק ק"נ ימים בכל כך חלקי' כמו הליט' שירויח הככר השנה | ||
|- | |- | ||
| | | | ||
| − | + | *{{#annot:days, for one liṭra|660|U8hO}}Example: one kikkar yields 9 liṭra a year and you want to know in how many days will one liṭra yield one pašuṭ? | |
| − | + | :<math>\scriptstyle\frac{\left(20\sdot12\right)\sdot9}{360\sdot100}=\frac{1}{X\sdot1}</math> | |
| − | + | |style="text-align:right;"|המשל בזה הככר ירויח ט' ליט' השנה ותרצה לידע בכמה ימים ירויח הליט' א"פ{{#annotend:U8hO}} | |
| − | |style="text-align:right;"|המשל בזה הככר ירויח ט' ליט' השנה ותרצה לידע בכמה ימים ירויח הליט' | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{X=\frac{150}{9}=16+\frac{2}{3}}}</math> | + | :Divide 150 days into 9 parts; the result is 16 days and 2-thirds. So, one liṭra yields one pašuṭ in 16 days and 2-thirds. |
| + | :<math>\scriptstyle{\color{blue}{X=\frac{150}{9}=16+\frac{2}{3}}}</math> | ||
|style="text-align:right;"|חלק ק"נ ימים בט' חלקי' שיבואו י"ו ימים וב' שלשי<br> | |style="text-align:right;"|חלק ק"נ ימים בט' חלקי' שיבואו י"ו ימים וב' שלשי<br> | ||
| − | הרי שבי"ו ימים וב' שלישי ירויח הליט' | + | הרי שבי"ו ימים וב' שלישי ירויח הליט' א"פ וכה"ל |
|- | |- | ||
| − | |||
| | | | ||
| − | + | :{{#annot:months, for some liṭra|660|LLGv}}28) If a man asks you: so and so liṭra yield so and so in a certain time. | |
| − | + | :In how much time will so and so liṭra yield the same? | |
| − | :{{#annot:months, for some liṭra|660|LLGv}}28) | ||
| − | :In how | ||
:<math>\scriptstyle\frac{a}{c\sdot b}=\frac{a}{X\sdot d}</math> | :<math>\scriptstyle\frac{a}{c\sdot b}=\frac{a}{X\sdot d}</math> | ||
| − | |style="text-align:right;"|כח | + | |style="text-align:right;"|כח <big>אם</big> ישאלך אדם כל כך ליט' ירויחו כל כך בכל כך זמן <s>כמה ליט'</s> <s>כמה</s> <sup>כל כך</sup> ליט' <s>בכל כך זמן</s> <sup>בכמה זמן</sup> ירויחו כהם <s>או בכל חדשים כמה ליט' ירויחו כל כך</s>{{#annotend:LLGv}} |
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> Multiply the number of liṭra by the number of months, then divide by the number of liṭra or months. | |
| + | :<math>\scriptstyle X=\frac{b\sdot c}{d}</math> | ||
|style="text-align:right;"|תרבה סכום הליט' כנגד סכום החדשים וחלק בכל כך חלקי' כמו שהם ליט' או חדשים | |style="text-align:right;"|תרבה סכום הליט' כנגד סכום החדשים וחלק בכל כך חלקי' כמו שהם ליט' או חדשים | ||
|- | |- | ||
| | | | ||
| − | + | *{{#annot:months, for some liṭra|660|XRzd}}Example: 50 liṭra yield 3 liṭra in 4 months. | |
| − | + | :In how much time will 75 liṭra yield the same? | |
| − | + | :<math>\scriptstyle\frac{3}{4\sdot50}=\frac{3}{X\sdot75}</math> | |
|style="text-align:right;"|המשל בזה נ' ליט' ירויחו ג' ליט' בד' חדשים<br> | |style="text-align:right;"|המשל בזה נ' ליט' ירויחו ג' ליט' בד' חדשים<br> | ||
הע"ה ליט' בכמה זמן ירויחו כהם{{#annotend:XRzd}} | הע"ה ליט' בכמה זמן ירויחו כהם{{#annotend:XRzd}} | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle{\color{blue}{x=\frac{50\sdot4}{75}=\frac{200}{75}=2+\frac{20}{30}}}</math> | + | :Multiply the number of liṭra by the number of months and say: 50 times 4 months; the result is 200 months. Divide them by 75; the result is 2 months and 20 days. We find that 75 liṭra yield in 2 months and 2 days as much as 50 liṭra yield in 4 months. |
| − | |style="text-align:right;"|הרבה סכום הליט' כנגד סכום החדשים ואמור נ' פעמי' ד' חדשים יבא ר' חדשים וחלקם בע"ה יבא ב' חדשים וכ' ימים נמצא שבב' חדשי' וב' ימים ירויחו הע"ה ליט' כל כך כמו הנ' ליט' ד' חדשים וכה | + | :<math>\scriptstyle{\color{blue}{x=\frac{50\sdot4}{75}=\frac{200}{75}=2+\frac{20}{30}}}</math> |
| + | |style="text-align:right;"|הרבה סכום הליט' כנגד סכום החדשים ואמור נ' פעמי' ד' חדשים יבא ר' חדשים וחלקם בע"ה יבא ב' חדשים וכ' ימים נמצא שבב' חדשי' וב' ימים ירויחו הע"ה ליט' כל כך כמו הנ' ליט' ד' חדשים וכה"ל | ||
|- | |- | ||
| − | |'''Find the Fund''' | + | |<span style=color:green>'''Find the Fund'''</span> |
| | | | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:liṭra for some months|666|gU7s}}29) | + | *{{#annot:liṭra for some months|666|gU7s}}29) If a man asks you: so and so liṭra yield so and so liṭra in a certain time. |
| − | :How many liṭra will yield the same in | + | :How many liṭra will yield the same in a certain? |
:<math>\scriptstyle\frac{a}{c\sdot b}=\frac{a}{d\sdot X}</math> | :<math>\scriptstyle\frac{a}{c\sdot b}=\frac{a}{d\sdot X}</math> | ||
| − | |style="text-align:right;"|כט | + | |style="text-align:right;"|כט <big>אם</big> ישאלך אדם כל כך ליט' ירויחו בכל כך זמן כל כך ליט‫'<br> |
כמה ליט' בכל כך זמן ירויחו כהם{{#annotend:gU7s}} | כמה ליט' בכל כך זמן ירויחו כהם{{#annotend:gU7s}} | ||
|- | |- | ||
| | | | ||
| − | : | + | :Multiply the number of the months by the number of the liṭra, then divide them by the number of the months or the liṭra we require. |
| − | + | :<math>\scriptstyle{\color{blue}{X=\frac{c\sdot b}{d}}}</math> | |
|style="text-align:right;"|תרבה סכום החדשים כנגד סכום הליט' וחלקם בכל כך חלקי' כמו החדשי' או הליט' שנרצה לידע | |style="text-align:right;"|תרבה סכום החדשים כנגד סכום הליט' וחלקם בכל כך חלקי' כמו החדשי' או הליט' שנרצה לידע | ||
|- | |- | ||
| | | | ||
| − | + | *{{#annot:liṭra for some months|666|EOv3}}Example: 25 liṭra yield 40 dinar in 6 months. | |
| − | + | :How many liṭra will yield the same in 8 months? | |
| − | + | :<math>\scriptstyle\frac{40}{6\sdot25}=\frac{40}{8\sdot X}</math> | |
|style="text-align:right;"|המשל בזה כ"ה ליט' ירויחו בו' חדשי' מ' דינרי‫'<br> | |style="text-align:right;"|המשל בזה כ"ה ליט' ירויחו בו' חדשי' מ' דינרי‫'<br> | ||
כמה ליט' ירויחו בהם בח' חדשים{{#annotend:EOv3}} | כמה ליט' ירויחו בהם בח' חדשים{{#annotend:EOv3}} | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{X=\frac{6\sdot25}{8}=\frac{150}{8}=18+\frac{15}{20}}}</math> | + | :Multiply the number of months by the number of the liṭra and say: 6 times 25 liṭra; the result is 150 liṭra. Divide them into 8 parts; the result is 18 liṭra and 15 dinar. We find that 18 liṭra and 15 dinar yield in 8 months 40 dinar, as 25 liṭra yield in 6 months. |
| − | + | :<math>\scriptstyle{\color{blue}{X=\frac{6\sdot25}{8}=\frac{150}{8}=18+\frac{15}{20}}}</math> | |
|style="text-align:right;"|תרבה סכום החדשים כנגד סכום הליט' ואמור ו' פעמי' כ"ה ליט' יבא ק"נ ליט‫'<br> | |style="text-align:right;"|תרבה סכום החדשים כנגד סכום הליט' ואמור ו' פעמי' כ"ה ליט' יבא ק"נ ליט‫'<br> | ||
חלקם בח' חלקי' יבא י"ח ליט' וט"ו דינרי‫'<br> | חלקם בח' חלקי' יבא י"ח ליט' וט"ו דינרי‫'<br> | ||
נמצא שי"ח ליט' וט"ו דינרי' ירויחו בח' חדשים מ' דינרי' כמו הכ"ה ליט' ו' חדשים | נמצא שי"ח ליט' וט"ו דינרי' ירויחו בח' חדשים מ' דינרי' כמו הכ"ה ליט' ו' חדשים | ||
|- | |- | ||
| − | |'''Find the Time''' | + | |<span style=color:green>'''Find the Time'''</span> |
| | | | ||
|- | |- | ||
| | | | ||
| − | :{{#annot:years, for some liṭra|660|ucM7}}30) I lent one liṭra for so and so | + | :{{#annot:years, for some liṭra|660|ucM7}}30) If a man asks you: I lent one liṭra for so and so a month. |
| − | :In how | + | :In how much time will so and so liṭra be doubled? |
:<math>\scriptstyle\frac{a}{1\sdot1}=\frac{\left(20\sdot12\right)\sdot b}{12\sdot X\sdot b}</math> | :<math>\scriptstyle\frac{a}{1\sdot1}=\frac{\left(20\sdot12\right)\sdot b}{12\sdot X\sdot b}</math> | ||
| − | |style="text-align:right;"|ל | + | |style="text-align:right;"|ל <big>אם</big> ישאלך אדם הלותי הליט' בכך וכך החדש<br> |
כל כך ליט' בכמה זמן יכפלו בלתי לשים ריוח אקרן{{#annotend:ucM7}} | כל כך ליט' בכמה זמן יכפלו בלתי לשים ריוח אקרן{{#annotend:ucM7}} | ||
|- | |- | ||
| | | | ||
| − | : | + | :Divide 20 year by the amount of money that a liṭra yields a month. |
| − | + | :<math>\scriptstyle{\color{blue}{X=\frac{20}{a}}}</math> | |
|style="text-align:right;"|חלק כ' שנים בכל כך חלקי' כמו המעות שירויח הליט' החדש | |style="text-align:right;"|חלק כ' שנים בכל כך חלקי' כמו המעות שירויח הליט' החדש | ||
|- | |- | ||
| | | | ||
| − | + | *{{#annot:years, for some liṭra|660|uGZJ}}Example: I lent one liṭra for 3 pešuṭim a month. | |
| − | + | :In how much time will 95 liṭra be doubled? | |
| − | + | :<math>\scriptstyle\frac{3}{1\sdot1}=\frac{\left(20\sdot12\right)\sdot95}{12\sdot X\sdot95}</math> | |
| − | |style="text-align:right;"|המשל בזה הלותי הליט' בגפ"ה הצ"ה ליט' בכמה זמן יכפלו{{#annotend:uGZJ}} | + | |style="text-align:right;"|המשל בזה הלותי הליט' בגפ"ה<br> |
| + | הצ"ה ליט' בכמה זמן יכפלו{{#annotend:uGZJ}} | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{X=\frac{20}{3}=6+\frac{8}{12}}}</math> | + | :Divide 20 years into 3 parts; the result is 6 years and 8 months. We find that 95 liṭra will be doubled in 6 years and 8 months. |
| − | + | :<math>\scriptstyle{\color{blue}{X=\frac{20}{3}=6+\frac{8}{12}}}</math> | |
|style="text-align:right;"|חלק כ' שנים בג' חלקי' שיבא ו' שנים וח' חדשי' נמצא שבו' שנים וח' חדשים יכפלו הצ"ה ליט‫' | |style="text-align:right;"|חלק כ' שנים בג' חלקי' שיבא ו' שנים וח' חדשי' נמצא שבו' שנים וח' חדשים יכפלו הצ"ה ליט‫' | ||
|- | |- | ||
| − | |||
| | | | ||
| − | + | :{{#annot:years, for some liṭra|660|9FcN}}31) If a man asks you: one kikkar yields so and so liṭra a year. | |
| − | + | :In how much time will so and so liṭra be doubled? | |
| − | :{{#annot:years, for some liṭra|660|9FcN}}31) | ||
| − | :In how | ||
:<math>\scriptstyle\frac{a}{1\sdot100}=\frac{b}{X\sdot b}</math> | :<math>\scriptstyle\frac{a}{1\sdot100}=\frac{b}{X\sdot b}</math> | ||
| − | |style="text-align:right;"|לא | + | |style="text-align:right;"|לא <big>אם</big> ישאלך אדם הככר ירויח השנה כל כך ליט‫' |
כל כך ליט' בכמה זמן יכפלו בלתי לשים ריוח אקרן{{#annotend:9FcN}} | כל כך ליט' בכמה זמן יכפלו בלתי לשים ריוח אקרן{{#annotend:9FcN}} | ||
|- | |- | ||
| | | | ||
| − | : | + | :Divide 100 years by the number of liṭra that the kikkar yields. |
| − | + | :<math>\scriptstyle{\color{blue}{X=\frac{100}{a}}}</math> | |
|style="text-align:right;"|חלק ק' שנים בכל כך חלקי' כמו הליט' שירויח הככר | |style="text-align:right;"|חלק ק' שנים בכל כך חלקי' כמו הליט' שירויח הככר | ||
|- | |- | ||
| | | | ||
| − | + | *{{#annot:years, for some liṭra|660|uvRl}}Example: one kikkar yields 8 liṭra a year. | |
| − | + | :In how much time will 45 liṭra be doubled? | |
| − | + | :<math>\scriptstyle\frac{8}{1\sdot100}=\frac{45}{X\sdot45}</math> | |
|style="text-align:right;"|המשל בזה הככר ירויח ח' ליט' השנה<br> | |style="text-align:right;"|המשל בזה הככר ירויח ח' ליט' השנה<br> | ||
המ"ה ליט' בכמה זמן יכפלו{{#annotend:uvRl}} | המ"ה ליט' בכמה זמן יכפלו{{#annotend:uvRl}} | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{X=\frac{100}{8}=12+\frac{6}{12}}}</math> | + | :Divide 100 years into 8 parts; the result is 12 years and a half. We find that 45 liṭra will be doubled in 12 years and a half. |
| − | + | :<math>\scriptstyle{\color{blue}{X=\frac{100}{8}=12+\frac{6}{12}}}</math> | |
|style="text-align:right;"|חלק ק' שנים בח' חלקי' שיבא י"ב שנים וחצי<br> | |style="text-align:right;"|חלק ק' שנים בח' חלקי' שיבא י"ב שנים וחצי<br> | ||
נמצא שבי"ב שנים וחצי יכפלו המ"ה ליט‫' | נמצא שבי"ב שנים וחצי יכפלו המ"ה ליט‫' | ||
|- | |- | ||
| − | |'''Pricing Problem - Find the Price''' | + | |<span style=color:green>'''Pricing Problem - Find the Price'''</span> |
| | | | ||
|- | |- | ||
| | | | ||
| − | :{{#annot:lint|629|Jc98}}32) | + | :{{#annot:lint|629|Jc98}}32) If a man asks you: one kantar [measurement of weight] of lint is worth 17 liṭra and 5 dinar. |
:One kantar is 165 liṭra. | :One kantar is 165 liṭra. | ||
:How much is one liṭra worth? | :How much is one liṭra worth? | ||
:<math>\scriptstyle\frac{165}{\left(20\sdot17\right)+5}=\frac{1}{X}</math> | :<math>\scriptstyle\frac{165}{\left(20\sdot17\right)+5}=\frac{1}{X}</math> | ||
| − | |style="text-align:right;"|לב | + | |style="text-align:right;"|לב <big>אם</big> ישאלך אדם ה{{#annot:kantar|1068|iyS4}}קנטרו'{{#annotend:iyS4}} מן המוך שוה י"ז ליט' וה' דינרי‫'<br> |
כמה ישוה הליט‫'<br> | כמה ישוה הליט‫'<br> | ||
והקנטרו' הוא קס"ה ליט‫'{{#annotend:Jc98}} | והקנטרו' הוא קס"ה ליט‫'{{#annotend:Jc98}} | ||
|- | |- | ||
| | | | ||
| − | : | + | :Divide the 17 liṭra and 5 dinar into 3 parts; each part is 5 liṭra and 15 dinar. |
| − | |style="text-align:right;"|חלק אלו הי"ז ליט' וה' דינרי' בג' חלקי' שיבא לכל חלק ה' ליט' וט"ו די‫' | + | |style="text-align:right;"|חלק אלו הי"ז ליט' וה' דינרי' בג' חלקי' שיבא לכל חלק ה' ליט' וט"ו די‫' |
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :Then, divide these 5 liṭra and 15 dinar into 5 parts; each part is 23 dinar. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אחרי כן חלק אלו הה' ליט' וט"ו די' בה' חלקי' שיבא לכל חלק כ"ג דינרי‫' |
|- | |- | ||
| − | |||
| | | | ||
| + | :Now, divide these 23 dinar into 11 parts; each part is 2 pešuṭim and 1 part of 11. We find that one liṭra of lint is worth 2 pešuṭim and 1 part of 11 of one pašuṭ. | ||
| + | :<math>\scriptstyle{\color{blue}{x=\frac{\frac{\frac{\left(20\sdot17\right)+5}{3}}{5}}{11}=\frac{\frac{\left(20\sdot5\right)+15}{5}}{11}=\frac{23}{11}=2+\frac{1}{11}}}</math> | ||
| + | |style="text-align:right;"|עת' תחלק אלו הכ"ג דינרי' בי"א חלקי' שיבא לכל חלק בה"פ וא' חלק מי"א<br> | ||
| + | נמצא שהליט' מן המוך שוה בה"פ וא' חלק מי"א בפשוט | ||
|- | |- | ||
| + | |<span style=color:green>'''Find the Earned Interest'''</span> | ||
| | | | ||
| − | :{{#annot:for one liṭra a month|638|nEKA}}33) | + | |- |
| − | :How | + | | |
| + | :{{#annot:for one liṭra a month|638|nEKA}}33) If a man asks you: so and so liṭra yield one pašuṭ a day. | ||
| + | :How much will one liṭra yield a month? | ||
:<math>\scriptstyle\frac{1}{1\sdot a}=\frac{X}{30\sdot1}</math> | :<math>\scriptstyle\frac{1}{1\sdot a}=\frac{X}{30\sdot1}</math> | ||
| − | |style="text-align:right;"|לג | + | |style="text-align:right;"|‫<ref>91r</ref>לג <big>אם</big> ישאלך אדם כל כך ליט' ירויחו היום א"פ<br> |
לאי זה חשבון יבא החדש הליט‫'{{#annotend:nEKA}} | לאי זה חשבון יבא החדש הליט‫'{{#annotend:nEKA}} | ||
|- | |- | ||
| | | | ||
| − | : | + | :Divide the liṭra by the number of liṭra that yield one pašuṭ a day. |
| − | + | :<math>\scriptstyle{\color{blue}{X=\frac{30}{a}}}</math> | |
| − | |style="text-align:right;"|חלק ליט' בכל כך חלקי' כמו הליט' שירויחו היום | + | |style="text-align:right;"|חלק ליט' בכל כך חלקי' כמו הליט' שירויחו היום א"פ |
|- | |- | ||
| | | | ||
| − | + | *{{#annot:for one liṭra a month|638|VBTh}}Example: 12 liṭra yield one pašuṭ a day and you want to know how much will one liṭra yield a month. | |
| − | + | :<math>\scriptstyle\frac{1}{1\sdot12}=\frac{X}{30\sdot1}</math> | |
| − | |||
|style="text-align:right;"|המשל בזה י"ב ליט' ירויחו היום א"פ<br> | |style="text-align:right;"|המשל בזה י"ב ליט' ירויחו היום א"פ<br> | ||
ותרצה לידע לאי זה חשבון יבא החדש הליט‫'{{#annotend:VBTh}} | ותרצה לידע לאי זה חשבון יבא החדש הליט‫'{{#annotend:VBTh}} | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{X=\frac{30}{12}=2+\frac{1}{2}}}</math> | + | :Divide 30 pešuṭim into 12 parts; the result is 2 pešuṭim and a half. We find that one liṭra yields 2 pešuṭim and a half a month. |
| + | :<math>\scriptstyle{\color{blue}{X=\frac{30}{12}=2+\frac{1}{2}}}</math> | ||
|style="text-align:right;"|חלק ל"פ בי"ב חלקי' שיבא ב"פ וחצי<br> | |style="text-align:right;"|חלק ל"פ בי"ב חלקי' שיבא ב"פ וחצי<br> | ||
| − | נמצא | + | נמצא שיבא החדש הליט' ב"פ וחצי וכה"ל |
|- | |- | ||
| | | | ||
| − | :{{#annot:for some liṭra a year|638|RDKN}}34) | + | :{{#annot:for some liṭra a year|638|RDKN}}34) If a man asks you: so and so liṭra yield one pašuṭ a day. |
:How many liṭra will 100 liṭra yield a year? | :How many liṭra will 100 liṭra yield a year? | ||
:<math>\scriptstyle\frac{1}{1\sdot a}=\frac{\left(20\sdot12\right)\sdot X}{360\sdot100}</math> | :<math>\scriptstyle\frac{1}{1\sdot a}=\frac{\left(20\sdot12\right)\sdot X}{360\sdot100}</math> | ||
| − | |style="text-align:right;"|לד | + | |style="text-align:right;"|לד <big>אם</big> ישאלך אדם כל כך ליט' ירויחו היום א"פ<br> |
כמה ליט' ירויחו ק' ליט' א' שנה{{#annotend:RDKN}} | כמה ליט' ירויחו ק' ליט' א' שנה{{#annotend:RDKN}} | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle x=\frac{150}{a}</math> | + | :Divide 150 by the number of liṭra that yield one pašuṭ a day. |
| − | |style="text-align:right;"|חלק ק"נ בכל כך חלקי' כמו הליט' שירויחו היום א"פ | + | :<math>\scriptstyle x=\frac{150}{a}</math> |
| + | |style="text-align:right;"|חלק ק"נ <sup>ליט'</sup> בכל כך חלקי' כמו הליט' שירויחו היום א"פ | ||
|- | |- | ||
| | | | ||
| − | + | *{{#annot:for some liṭra a year|638|Ins5}}Example: 15 liṭra yield one pašuṭ a day. | |
| − | + | :How much will 100 liṭra yield a year? | |
| − | + | :<math>\scriptstyle\frac{1}{1\sdot15}=\frac{\left(20\sdot12\right)\sdot X}{360\sdot100}</math> | |
|style="text-align:right;"|המשל בזה ט"ו ליט' ירויחו היום א"פ<br> | |style="text-align:right;"|המשל בזה ט"ו ליט' ירויחו היום א"פ<br> | ||
כמה ירויחו ק' ליט' א' שנה‫'{{#annotend:Ins5}} | כמה ירויחו ק' ליט' א' שנה‫'{{#annotend:Ins5}} | ||
|- | |- | ||
| | | | ||
| − | : | + | :Divide 150 liṭra into 15 parts; the result is 10 liṭra. We find that 100 liṭra yield 10 liṭra a year. |
| + | :<math>\scriptstyle{\color{blue}{x=\frac{150}{15}=10}}</math> | ||
|style="text-align:right;"|חלק ק"נ ליט' בט"ו חלקי' שיבא י' ליט‫'<br> | |style="text-align:right;"|חלק ק"נ ליט' בט"ו חלקי' שיבא י' ליט‫'<br> | ||
נמצא שק' ליט' ירויחו א' שנה י' ליט‫' | נמצא שק' ליט' ירויחו א' שנה י' ליט‫' | ||
|- | |- | ||
| | | | ||
| − | :{{#annot:liṭra for some months|666|nqXZ}}35) | + | :{{#annot:liṭra for some months|666|nqXZ}}35) If a man asks you: one kikkar yields 29 liṭra, 13 dinar and 7 pešuṭim a year. |
:How many liṭra will yield the same in 7 months? | :How many liṭra will yield the same in 7 months? | ||
:<math>\scriptstyle\frac{29+\frac{13}{20}+\frac{7}{12\sdot20}}{12\sdot100}=\frac{29+\frac{13}{20}+\frac{7}{12\sdot20}}{7\sdot X}</math> | :<math>\scriptstyle\frac{29+\frac{13}{20}+\frac{7}{12\sdot20}}{12\sdot100}=\frac{29+\frac{13}{20}+\frac{7}{12\sdot20}}{7\sdot X}</math> | ||
| − | |style="text-align:right;"|לה) אם ישאלך אדם הככר ירויח השנה כ"ט ליט' וי"ג דינרי' וז"פ<br> | + | |style="text-align:right;"|לה) <big>אם</big> ישאלך אדם הככר ירויח השנה כ"ט ליט' וי"ג דינרי' וז"פ<br> |
כמה ליט' ירויחו בהם ז' חדשי‫'{{#annotend:nqXZ}} | כמה ליט' ירויחו בהם ז' חדשי‫'{{#annotend:nqXZ}} | ||
|- | |- | ||
| | | | ||
| − | : | + | :Multiply the months of the year by the number of the liṭra, i.e. 100 liṭra, then divide them by the number of the months we require, i.e. 7 months. |
| − | |||
|style="text-align:right;"|תרבה חדשי השנה עם סכום הליט' דהיינו ק' ליט‫'<br> | |style="text-align:right;"|תרבה חדשי השנה עם סכום הליט' דהיינו ק' ליט‫'<br> | ||
| − | וחלקם בכל כך חלקי' כמו החדשי' שנרצה לידע דהיינו הז' חדשים | + | וחלקם בכל כך חלקי' כמו החדשי' שנרצה לידע דהיינו הז' חדשים |
| − | ואמור י"ב פעמי' ק' ליט' יבא אלף ור‫' | + | |- |
| − | + | | | |
| − | + | :Say: 12 times 100 liṭra; the result is 1200. | |
| + | |style="text-align:right;"|ואמור י"ב פעמי' ק' ליט' יבא אלף ור‫' | ||
|- | |- | ||
| − | |||
| | | | ||
| + | :Divide them by 7; the result is 171 liṭra, 8 dinar, a half and 6 parts of 7 of one pašuṭ. | ||
| + | :<math>\scriptstyle{\color{blue}{X=\frac{12\sdot100}{7}=\frac{1200}{7}=171+\frac{8}{20}+\frac{6+\frac{6}{7}}{20\sdot12}}}</math> | ||
| + | |style="text-align:right;"|חלקם על ז' שיבא קע"א ליט' וח' דינרי' וחצי וו' חלקי' מז' בפשוט | ||
|- | |- | ||
| | | | ||
| − | :{{#annot:years, for one kikkar|660|oMv3}}36) | + | :We find that 171 liṭra, 8 dinar, a half and 6 parts of 7 of one pašuṭ yield in 7 months as one kikkar yields a year. |
| − | :In how | + | |style="text-align:right;"|נמצא שקע"א ליט' וח' די' וחצי וו' חלקי' מז' בפשוט ירויחו כ"כ בז' חדשי' כמו הככר א' שנה וכה"ל |
| + | |- | ||
| + | |<span style=color:green>'''Find the Time'''</span> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :{{#annot:years, for one kikkar|660|oMv3}}36) If a man asks you: one kikkar yields so and so liṭra a year. | ||
| + | :In how much time it will be doubled? | ||
:<math>\scriptstyle\frac{a}{1\sdot1}=\frac{100}{X\sdot1}</math> | :<math>\scriptstyle\frac{a}{1\sdot1}=\frac{100}{X\sdot1}</math> | ||
| − | |style="text-align:right;"|לו | + | |style="text-align:right;"|לו <big>אי"א</big> הככר ירויח כ"כ ליט' השנה<br> |
בכמה זמן יכפלו בלתי לשים ריוח אקרן{{#annotend:oMv3}} | בכמה זמן יכפלו בלתי לשים ריוח אקרן{{#annotend:oMv3}} | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle x=\frac{100}{a}</math> | + | :Divide 100 years by the number of liṭra that the kikkar yields a year. |
| + | :<math>\scriptstyle x=\frac{100}{a}</math> | ||
|style="text-align:right;"|חלק ק' שנים בכל כך חלקי' כמו הליט' שירויח הככר השנה | |style="text-align:right;"|חלק ק' שנים בכל כך חלקי' כמו הליט' שירויח הככר השנה | ||
|- | |- | ||
| | | | ||
| − | + | *{{#annot:years, for one kikkar|660|B1s0}}Example: one kikkar yields 14 liṭra a year and you want to know in how much time it will be doubled? | |
| − | + | :<math>\scriptstyle\frac{14}{1\sdot1}=\frac{100}{X\sdot1}</math> | |
| − | |||
|style="text-align:right;"|המשל בזה הככר ירויח י"ד ליט' השנה ותרצה לידע בכמה זמן יכפלו{{#annotend:B1s0}} | |style="text-align:right;"|המשל בזה הככר ירויח י"ד ליט' השנה ותרצה לידע בכמה זמן יכפלו{{#annotend:B1s0}} | ||
|- | |- | ||
| | | | ||
| − | : | + | :Divide 100 years into 14 parts; the result is 7 years, 1 month, 21 days and 3 parts of 7 of a day. We find that it will be doubled in 7 years, 1 month, 21 days and 3 parts of 7 of a day. |
| − | + | :<math>\scriptstyle{\color{blue}{x=\frac{100}{14}=7+\frac{1}{12}+\frac{21+\frac{3}{7}}{12\sdot30}}}</math> | |
|style="text-align:right;"|חלק ק' שנים בי"ד חלקי' שיבא ז' שנים וא' חדש וכ"א ימים וג' חלקי' מז' ביום<br> | |style="text-align:right;"|חלק ק' שנים בי"ד חלקי' שיבא ז' שנים וא' חדש וכ"א ימים וג' חלקי' מז' ביום<br> | ||
נמצא שבז' שנים וא' חדש וכ"א ימים וג' חלקי' מז' ביום יכפלו | נמצא שבז' שנים וא' חדש וכ"א ימים וג' חלקי' מז' ביום יכפלו | ||
|- | |- | ||
| | | | ||
| − | :{{#annot:years, for some dinar|660|c2DO}}37) | + | :{{#annot:years, for some dinar|660|c2DO}}37) If a man asks you: I lent a liṭra at 2 pešiṭim and a half a month. |
| − | :In how | + | :In how much time it will be doubled? |
:<math>\scriptstyle\frac{2+\frac{1}{2}}{1\sdot20}=\frac{12\sdot a}{X\sdot12\sdot a}</math> | :<math>\scriptstyle\frac{2+\frac{1}{2}}{1\sdot20}=\frac{12\sdot a}{X\sdot12\sdot a}</math> | ||
| − | |style="text-align:right;"|לז | + | |style="text-align:right;"|לז <big>אי"א</big> הלויתי הליט' לחשבון ב"פ וחצי החדש<br> |
בכמה זמן יכפלו בלתי לשים רבית אקרן{{#annotend:c2DO}} | בכמה זמן יכפלו בלתי לשים רבית אקרן{{#annotend:c2DO}} | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{X=\frac{20}{2+\frac{1}{2}}}}</math> | + | :Divide 20 years by the amount of money that the liṭra yields a month. |
| − | |style="text-align:right;"|חלק כ' שנים בכל כך חלקים כמו המעות שירויח הליט' החדש | + | :<math>\scriptstyle{\color{blue}{X=\frac{20}{2+\frac{1}{2}}}}</math> |
| + | |style="text-align:right;"|חלק כ' שנים בכל כך חלקים כמו המעות שירויח הליט' החדש | ||
|- | |- | ||
| | | | ||
| − | + | *{{#annot:years, for some dinar|660|hv2h}}Example: one liṭra yields 2 pešiṭim and a half a month and you want to know in how much time it will be doubled. | |
| − | + | :<math>\scriptstyle\frac{2+\frac{1}{2}}{1\sdot20}=\frac{12\sdot20}{X\sdot12\sdot20}</math> | |
| − | + | |style="text-align:right;"|המ"ב הלותי כ' דינרי' לחשבון ב"פ וחצי החדש <sup>הליט'</sup> ותרצה לידע בכמה זמן יכפלו{{#annotend:hv2h}} | |
| − | |style="text-align:right;"|הלותי כ' דינרי' לחשבון ב"פ וחצי החדש הליט' ותרצה לידע בכמה זמן יכפלו{{#annotend:hv2h}} | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{X=\frac{20}{2+\frac{1}{2}}=8}}</math> | + | :Divide 20 years by 2 and a half; the result is 8 years. We find that it will be doubled in 8 years. |
| + | :<math>\scriptstyle{\color{blue}{X=\frac{20}{2+\frac{1}{2}}=8}}</math> | ||
|style="text-align:right;"|חלק כ' שני' בב' וחצי שיבא ח' שני‫'<br> | |style="text-align:right;"|חלק כ' שני' בב' וחצי שיבא ח' שני‫'<br> | ||
| − | נמצא שבח' שנים יכפלו וכה | + | נמצא שבח' שנים יכפלו וכה"ל |
|- | |- | ||
| − | |'''Find the Earned Interest''' | + | |<span style=color:green>'''Find the Earned Interest'''</span> |
| | | | ||
|- | |- | ||
| | | | ||
| − | :{{#annot:liṭra for one day|666|UlEj}}38) One kikkar yields so and so liṭra a year. | + | :{{#annot:liṭra for one day|666|UlEj}}38) If a man asks you: One kikkar yields so and so liṭra a year. |
:How many liṭra will yield one pašuṭ a day? | :How many liṭra will yield one pašuṭ a day? | ||
:<math>\scriptstyle\frac{\left(20\sdot12\right)\sdot a}{360\sdot100}=\frac{1}{1\sdot X}</math> | :<math>\scriptstyle\frac{\left(20\sdot12\right)\sdot a}{360\sdot100}=\frac{1}{1\sdot X}</math> | ||
| − | |style="text-align:right;"|לח | + | |style="text-align:right;"|לח <big>אם</big> ישאלך אדם הככר ירויח כ"כ ליט' השנה<br> |
כמה ליט' ירויחו היום א"פ{{#annotend:UlEj}} | כמה ליט' ירויחו היום א"פ{{#annotend:UlEj}} | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle x=\frac{150}{a}</math> | + | :Divide 150 liṭra by the [interest]. |
| + | :<math>\scriptstyle x=\frac{150}{a}</math> | ||
|style="text-align:right;"|חלק ק"נ ליט' בכל כך חלקי‫' | |style="text-align:right;"|חלק ק"נ ליט' בכל כך חלקי‫' | ||
|- | |- | ||
| | | | ||
| − | + | *{{#annot:liṭra for one day|666|wNgJ}}Such as, one kikkar yields 13 liṭra a year and you want to know how many liṭra will yield one pašuṭ a day. | |
| − | + | :<math>\scriptstyle\frac{\left(20\sdot12\right)\sdot13}{360\sdot100}=\frac{1}{1\sdot X}</math> | |
| − | |||
|style="text-align:right;"|כמו הליט' שירויחו הככר השנה הב' הככר ירויח י"ג ליט' השנה ותרצה לידע כמה ליט' ירויחו היום א"פ{{#annotend:wNgJ}} | |style="text-align:right;"|כמו הליט' שירויחו הככר השנה הב' הככר ירויח י"ג ליט' השנה ותרצה לידע כמה ליט' ירויחו היום א"פ{{#annotend:wNgJ}} | ||
|- | |- | ||
| | | | ||
| − | : | + | :Divide 150 liṭra into 13 parts; the result is 11 liṭra, 10 dinar, 9 pešuṭim and 3 parts of 13 of one pašuṭ; and this amount yields one pašuṭ a day. |
| − | + | :<math>\scriptstyle{\color{blue}{x=\frac{150}{13}=11+\frac{10}{20}+\frac{9+\frac{3}{13}}{12\sdot20}}}</math> | |
|style="text-align:right;"|חלק ק"נ ליט' בי"ג חלקי' שיבא י"א ליט' וי' די' וט"פ וג' חלקי' מי"ג בפשוט וכל כך מעות ירויחו היום א"פ | |style="text-align:right;"|חלק ק"נ ליט' בי"ג חלקי' שיבא י"א ליט' וי' די' וט"פ וג' חלקי' מי"ג בפשוט וכל כך מעות ירויחו היום א"פ | ||
|- | |- | ||
| | | | ||
| − | :{{#annot:for some liṭra a day|638|jYdL}}39) | + | :{{#annot:for some liṭra a day|638|jYdL}}39) If a man asks you: I lent a liṭra for so and so a month. |
:How much will 100 liṭra yield a day? | :How much will 100 liṭra yield a day? | ||
:<math>\scriptstyle\frac{a}{30\sdot1}=\frac{X}{1\sdot100}</math> | :<math>\scriptstyle\frac{a}{30\sdot1}=\frac{X}{1\sdot100}</math> | ||
| − | |style="text-align:right;"|לט | + | |style="text-align:right;"|לט <big>אי"א</big> הלותי הליט' החדש לחשבון כך וכך<br> |
כמה ירויחו היום ק' ליט‫'{{#annotend:jYdL}} | כמה ירויחו היום ק' ליט‫'{{#annotend:jYdL}} | ||
|- | |- | ||
| | | | ||
| − | : | + | :Multiply 3 and a third by the interest. |
| − | + | :<math>\scriptstyle{\color{blue}{X=\left(3+\frac{1}{3}\right)\sdot a}}</math> | |
|style="text-align:right;"|תרבה ג' ושליש כנגד מעות הרבית | |style="text-align:right;"|תרבה ג' ושליש כנגד מעות הרבית | ||
|- | |- | ||
| | | | ||
| − | + | *{{#annot:for some liṭra a day|638|0TuA}}Example: I lent a liṭra for 3 pešiṭim a month and you want to know how much will 100 liṭra yield a day? | |
| − | + | :<math>\scriptstyle\frac{3}{30\sdot1}=\frac{X}{1\sdot100}</math> | |
| − | |||
|style="text-align:right;"|המשל בזה הלותי הליט' לחשבון ג"פ החדש<br> | |style="text-align:right;"|המשל בזה הלותי הליט' לחשבון ג"פ החדש<br> | ||
ותרצה לידע כמה ירויחו היום ק' ליט‫'{{#annotend:0TuA}} | ותרצה לידע כמה ירויחו היום ק' ליט‫'{{#annotend:0TuA}} | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{X=\left(3+\frac{1}{3}\right)\sdot3=10}}</math> | + | :Multiply 3 and a third by 3 and say: 3 times and a third by 3 are 10 pešuṭim. We find that 100 liṭra yield 10 pešuṭim a day. |
| + | :<math>\scriptstyle{\color{blue}{X=\left(3+\frac{1}{3}\right)\sdot3=10}}</math> | ||
|style="text-align:right;"|תרבה ג' ושליש עם ג' ואמור ג' פעמי' ושליש ג' י"פ<br> | |style="text-align:right;"|תרבה ג' ושליש עם ג' ואמור ג' פעמי' ושליש ג' י"פ<br> | ||
| − | נמצא שק' ליט' ירויחו היום י"פ וכה | + | נמצא שק' ליט' ירויחו היום י"פ וכה"ל |
|- | |- | ||
| | | | ||
| − | :{{#annot:for one kikkar a year|638|cQe2}}40) One kikkar yields so and so pešuṭim a day. | + | :{{#annot:for one kikkar a year|638|cQe2}}40) If a man asks you: One kikkar yields so and so pešuṭim a day. |
| − | :How | + | :How much will one kikkar yield a year? |
:<math>\scriptstyle\frac{a}{1\sdot1}=\frac{\left(20\sdot12\right)\sdot X}{360\sdot1}</math> | :<math>\scriptstyle\frac{a}{1\sdot1}=\frac{\left(20\sdot12\right)\sdot X}{360\sdot1}</math> | ||
| − | |style="text-align:right;"|מ | + | |style="text-align:right;"|מ <big>אם</big> ישאלך אדם הככר ירויח כ"כ פשוטי' היום<br> |
כמה ירויח הככר השנה{{#annotend:cQe2}} | כמה ירויח הככר השנה{{#annotend:cQe2}} | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle x=a\sdot\left(1+\frac{1}{2}\right)</math> | + | :Multiply 1 and a half by the money that the kikkar yields a day. |
| − | |style="text-align:right;"|תרבה א' וחצי עם המעות שירויח הככר היום | + | :<math>\scriptstyle x=a\sdot\left(1+\frac{1}{2}\right)</math> |
| + | |style="text-align:right;"|תרבה א' וחצי ‫<ref>91v</ref>עם המעות שירויח הככר היום | ||
|- | |- | ||
| | | | ||
| − | + | *{{#annot:for one kikkar a year|638|eMAO}}Example: one kikkar yields 10 pešuṭim a day and you want to know how much will it yield a year? | |
| − | + | :<math>\scriptstyle\frac{10}{1\sdot1}=\frac{\left(20\sdot12\right)\sdot X}{360\sdot1}</math> | |
| − | |||
|style="text-align:right;"|המשל בזה הככר ירויח היום י"פ ותרצה לידע כמה יבוא השנה{{#annotend:eMAO}} | |style="text-align:right;"|המשל בזה הככר ירויח היום י"פ ותרצה לידע כמה יבוא השנה{{#annotend:eMAO}} | ||
|- | |- | ||
| | | | ||
| − | :::<math>\scriptstyle{\color{blue}{x=10\sdot\left(1+\frac{1}{2}\right)=15}}</math> | + | :Multiply 1 and a half by 10 and say: 1 and a half times 10 liṭra are 15 liṭra. We find that the kikkar yields 15 liṭra a year. |
| + | :<math>\scriptstyle{\color{blue}{x=10\sdot\left(1+\frac{1}{2}\right)=15}}</math> | ||
|style="text-align:right;"|תרבה א' וחצי עם י' ואמור א' וחצי פעמי' י' ליט' יבא ט"ו ליט‫'<br> | |style="text-align:right;"|תרבה א' וחצי עם י' ואמור א' וחצי פעמי' י' ליט' יבא ט"ו ליט‫'<br> | ||
נמצא שירויח הככר ט"ו ליט' השנה | נמצא שירויח הככר ט"ו ליט' השנה | ||
|- | |- | ||
| | | | ||
| − | :{{#annot:for one liṭra a month|638|6SAa}}41) One kikkar yields so and so pešuṭim a day | + | :{{#annot:for one liṭra a month|638|6SAa}}41) If a man asks you: One kikkar yields so and so pešuṭim a day, how much will one liṭra yield a month? |
| − | |||
:<math>\scriptstyle\frac{a}{1\sdot100}=\frac{X}{30\sdot1}</math> | :<math>\scriptstyle\frac{a}{1\sdot100}=\frac{X}{30\sdot1}</math> | ||
| − | |style="text-align:right;"|מא | + | |style="text-align:right;"|מא <big>אם</big> ישאלך אדם הככר ירויח היום כ"כ פשוטי‫'<br> |
כמה יבא החדש הליט‫'{{#annotend:6SAa}} | כמה יבא החדש הליט‫'{{#annotend:6SAa}} | ||
|- | |- | ||
| | | | ||
| + | :Divide the money that the kikkar earns a day by 3 and a third. | ||
:<math>\scriptstyle x=\frac{a}{3+\frac{1}{3}}</math> | :<math>\scriptstyle x=\frac{a}{3+\frac{1}{3}}</math> | ||
| − | |style="text-align:right;"|חלק בג' ושליש | + | |style="text-align:right;"|חלק בג' ושליש המעות שירויח הככר היום |
|- | |- | ||
| | | | ||
| − | + | *{{#annot:for one liṭra a month|638|N3Io}}Example: one kikkar yields 10 pešuṭim a day and you want to know how much will one liṭra yield a month? | |
| − | + | :<math>\scriptstyle\frac{10}{1\sdot100}=\frac{X}{30\sdot1}</math> | |
| − | |||
|style="text-align:right;"|המשל בזה הככר ירויח היום י"פ ותרצה לידע כמה יבא החדש הליט‫'{{#annotend:N3Io}} | |style="text-align:right;"|המשל בזה הככר ירויח היום י"פ ותרצה לידע כמה יבא החדש הליט‫'{{#annotend:N3Io}} | ||
|- | |- | ||
| | | | ||
| − | : | + | :Divide 10 pešuṭim by 3 and a third; the result is 3 pešuṭim. We find that one liṭra yields 3 pešuṭim a month. |
| + | :<math>\scriptstyle{\color{blue}{x=\frac{10}{3+\frac{1}{3}}=3}}</math> | ||
|style="text-align:right;"|חלק י"פ בג' ושליש יבא ג"פ נמצא שיבא לחשבון גפה"ה וכה"ל | |style="text-align:right;"|חלק י"פ בג' ושליש יבא ג"פ נמצא שיבא לחשבון גפה"ה וכה"ל | ||
|- | |- | ||
| | | | ||
| − | :{{#annot:for one liṭra a month|638|nbZq}}42) One kikkar yields so and so liṭra a year. | + | :{{#annot:for one liṭra a month|638|nbZq}}42) If a man asks you: One kikkar yields so and so liṭra a year. |
:How much will one liṭra yield a month? | :How much will one liṭra yield a month? | ||
:<math>\scriptstyle\frac{\left(20\sdot12\right)\sdot a}{12\sdot100}=\frac{X}{1\sdot1}</math> | :<math>\scriptstyle\frac{\left(20\sdot12\right)\sdot a}{12\sdot100}=\frac{X}{1\sdot1}</math> | ||
| − | |style="text-align:right;"|מב | + | |style="text-align:right;"|מב <big>אי"א</big> הככר ירויח כל כך ליט' השנה<br> |
כמה יבא החדש הליט‫'{{#annotend:nbZq}} | כמה יבא החדש הליט‫'{{#annotend:nbZq}} | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle x=\frac{a}{5}</math> | + | :Divide the [number of] liṭra the kikkar earns a year by five and consider them as pešuṭim. |
| + | :<math>\scriptstyle x=\frac{a}{5}</math> | ||
|style="text-align:right;"|חלק לחומש הליט' שירויח הככר השנה ותחשבם פשוט‫' | |style="text-align:right;"|חלק לחומש הליט' שירויח הככר השנה ותחשבם פשוט‫' | ||
|- | |- | ||
| | | | ||
| − | + | *{{#annot:for one liṭra a month|638|Os8m}}Example: one kikkar yields 12 liṭra a year and you want to know how much will one liṭra yield a month? | |
| − | + | :<math>\scriptstyle\frac{\left(20\sdot12\right)\sdot12}{12\sdot100}=\frac{X}{1\sdot1}</math> | |
| − | |||
|style="text-align:right;"|המשל בזה הככר ירויח י"ב ליט' השנה ותרצה לידע כמה יבא החדש הליט‫'{{#annotend:Os8m}} | |style="text-align:right;"|המשל בזה הככר ירויח י"ב ליט' השנה ותרצה לידע כמה יבא החדש הליט‫'{{#annotend:Os8m}} | ||
|- | |- | ||
| | | | ||
| − | : | + | :Divide 12 liṭra by 5 and consider them as pešuṭim; the result is 2 pešuṭim and 2-fifths of a pašuṭ. |
| − | |style="text-align:right;"|חלק י"ב ליט' ה' לחומש ותחשבם פשוטי' שיבא ב"פ וב' חמשי פשוט נמצא שיבא החדש הליט' ב"פ וב' חמשי' וכה"ל | + | :<math>\scriptstyle{\color{blue}{x=\frac{12}{5}=2+\frac{2}{5}}}</math> |
| + | |style="text-align:right;"|חלק י"ב ליט' <s>ה'</s> לחומש ותחשבם פשוטי' שיבא ב"פ וב' חמשי פשוט | ||
| + | |- | ||
| + | | | ||
| + | :We find that the liṭra yields 2 pešuṭim and 2-fifths a month. | ||
| + | |style="text-align:right;"|נמצא שיבא החדש הליט' ב"פ וב' חמשי' וכה"ל | ||
|- | |- | ||
| | | | ||
| − | :{{#annot:for some liṭra a month|638|GL9C}}43) | + | :{{#annot:for some liṭra a month|638|GL9C}}43) If you lend 100 liṭra for one month at 8 liṭra a year and you want to know how much will be earned in a short way. |
| − | |||
:<math>\scriptstyle\frac{8}{12\sdot100}=\frac{X}{1\sdot100}</math> | :<math>\scriptstyle\frac{8}{12\sdot100}=\frac{X}{1\sdot100}</math> | ||
| − | |style="text-align:right;"|מג | + | |style="text-align:right;"|מג <big>אם</big> תלוה ק' ליט' א' חדש לחשבון ח' ליט' השנה ותרצה לידע כמה יבא בדרך קצרה{{#annotend:GL9C}} |
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle\frac{8}{12\sdot100}=\frac{a}{1\sdot100}</math> | + | :Follow this way and say: if you loan them for one month at 8 liṭra a year, the profit would be 100 pešuṭim, which are 8 dinar and 4 pešuṭim. |
| − | |style="text-align:right;"|כלך לדרך זו ואמור אם היית מלוה אותם א' חדש לחשבון ח' ליט' השנה | + | :<math>\scriptstyle\frac{8}{12\sdot100}=\frac{a}{1\sdot100}</math> |
| + | |style="text-align:right;"|כלך לדרך זו ואמור אם היית מלוה אותם א' חדש לחשבון ח' ליט' השנה היה עולה הריוח ק' פשוטי' שהם ח' די' וד"פ | ||
|- | |- | ||
| | | | ||
| − | : | + | :So, each liṭra yields 1 pašuṭ a month. |
| − | + | :<math>\scriptstyle{\color{blue}{a=\frac{100}{12\sdot20}=\frac{8}{20}+\frac{4}{12\sdot20}}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|שיבא לכל ליט' א"פ בחדש |
|- | |- | ||
| | | | ||
| − | : | + | :When you add 8 additional liṭra, the profit becomes 1 pašuṭ and 3-fifths of a pašuṭ a month for each liṭra. |
| − | + | :<math>\scriptstyle{\color{blue}{\frac{8}{12\sdot100}=\frac{1+\frac{3}{5}}{12\sdot20}}}</math> | |
|style="text-align:right;"|וכשאתה מוסיף עוד ח' ליט' הרי שעולה הריוח בכל ליט' בא' חדש א' פשוט וג' חמשי פשוט | |style="text-align:right;"|וכשאתה מוסיף עוד ח' ליט' הרי שעולה הריוח בכל ליט' בא' חדש א' פשוט וג' חמשי פשוט | ||
|- | |- | ||
| | | | ||
| − | : | + | :Then, one kikkar yields 160 pešuṭim that are a total of 13 dinar and 4 pešuṭim a month. |
| − | |||
|style="text-align:right;"|שיבא הככר ק' פשוטי' וס' חומשי פשוט שהוא סך הכל י"ג די' וד"פ וכך יבא הככר החדש | |style="text-align:right;"|שיבא הככר ק' פשוטי' וס' חומשי פשוט שהוא סך הכל י"ג די' וד"פ וכך יבא הככר החדש | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | :<math>\scriptstyle{\color{blue}{x=100\sdot\frac{8}{12\sdot100}=100\sdot\frac{1+\frac{3}{5}}{12\sdot20}=\frac{100+60}{12\sdot20}=\frac{13}{20}+\frac{4}{12\sdot20}}}</math> | ||
|- | |- | ||
| | | | ||
| − | + | *At 7 liṭra a year | |
| − | + | :<math>\scriptstyle\frac{7}{12\sdot100}=\frac{X}{1\sdot100}</math> | |
|style="text-align:right;"|ולחש' ז' ליט' השנה | |style="text-align:right;"|ולחש' ז' ליט' השנה | ||
|- | |- | ||
| | | | ||
| − | : | + | :Each liṭra yields 1 pašuṭ and 2-fifths of a pašuṭ per month. |
| − | + | :<math>\scriptstyle{\color{blue}{\frac{7}{12\sdot100}=\frac{1+\frac{2}{5}}{12\sdot20}}}</math> | |
|style="text-align:right;"|יבא לכל ליט' א"פ וב' חומשי פשוט בחדש | |style="text-align:right;"|יבא לכל ליט' א"פ וב' חומשי פשוט בחדש | ||
|- | |- | ||
| | | | ||
| − | : | + | :So, one kikkar yields 12 dinar minus 4 pešuṭim a month. |
| − | + | :<math>\scriptstyle{\color{blue}{x=100\sdot\frac{7}{12\sdot100}=100\sdot\frac{1+\frac{2}{5}}{12\sdot20}=\frac{12}{20}-\frac{4}{12\sdot20}}}</math> | |
| − | |style="text-align:right;"|שיבא הככר בחדש י"ב די' פד"פ | + | |style="text-align:right;"|שיבא הככר בחדש י"ב די' פד"פ <sup>פחות ד' פשוטי'</sup> וכה"ל |
|- | |- | ||
| | | | ||
| − | * | + | *Another [method] for the question written above: |
|style="text-align:right;"|אחר על זאת השאלה הכתובה למעלה | |style="text-align:right;"|אחר על זאת השאלה הכתובה למעלה | ||
|- | |- | ||
| | | | ||
| − | :You lend 100 liṭra for one month at 8 liṭra a year | + | :If You lend 100 liṭra for one month at 8 liṭra a year, and you want to know how much will be earned in a short way. |
| − | |||
:<math>\scriptstyle\frac{8}{12\sdot100}=\frac{X}{1\sdot100}</math> | :<math>\scriptstyle\frac{8}{12\sdot100}=\frac{X}{1\sdot100}</math> | ||
|style="text-align:right;"|אם תלוה ק' ליט' א' חדש לחשבון ח' ליט' השנה ותרצה לידע כמה יבא בדרך קצרה | |style="text-align:right;"|אם תלוה ק' ליט' א' חדש לחשבון ח' ליט' השנה ותרצה לידע כמה יבא בדרך קצרה | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle\frac{\frac{20}{20}}{12\sdot100}=\frac{a}{1\sdot100}</math> | + | :Follow this way and say: if you lend 100 liṭra for one month at 20 dinar a year. |
| + | :<math>\scriptstyle\frac{\frac{20}{20}}{12\sdot100}=\frac{a}{1\sdot100}</math> | ||
|style="text-align:right;"|כלך לדרך זו ואמור אם היית מלוה ק' ליט' א' חדש לחשבון כ' די' השנה | |style="text-align:right;"|כלך לדרך זו ואמור אם היית מלוה ק' ליט' א' חדש לחשבון כ' די' השנה | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{a=\frac{20}{12\sdot20}=\frac{\frac{5}{3}}{20}}}</math> | + | :It yields 20 pešuṭim a month, which are 5 yadot ([one yadot] is a third of a dinar). |
| − | + | :<math>\scriptstyle{\color{blue}{a=\frac{20}{12\sdot20}=\frac{\frac{5}{3}}{20}}}</math> | |
| − | + | |style="text-align:right;"|יבא החדש כ"פ שהם ה' ידות <sup>שלישיון דינר</sup> | |
| − | |style="text-align:right;"|יבא החדש כ"פ שהם ה' ידות | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{x=8\sdot\frac{\frac{5}{3}}{20}=\frac{\frac{40}{3}}{20}=\frac{13}{20}+\frac{4}{12\sdot20}}}</math> | + | :Say: 5 times 8 yadot (8 liṭra) are 40 yadot, which are 13 dinar and 4 pešuṭim. |
| − | + | :<math>\scriptstyle{\color{blue}{x=8\sdot\frac{\frac{5}{3}}{20}=\frac{\frac{40}{3}}{20}=\frac{13}{20}+\frac{4}{12\sdot20}}}</math> | |
| − | |style="text-align:right;"|ואמור ה' פעמי' ח' ידות | + | |style="text-align:right;"|ואמור ה' פעמי' ח' ידות <sup>ח' ליט'</sup> הם מ' ידות <sup>שלישיון</sup> שהם י"ג די' וד"פ |
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle\frac{7}{12\sdot100}=\frac{X}{1\sdot100}</math> | + | :According to this calculation, say also: 5 times 7 yadot are 35 yadot, which are 12 dinar minus 4 pešuṭim. |
| − | + | :<math>\scriptstyle\frac{7}{12\sdot100}=\frac{X}{1\sdot100}</math> | |
| − | + | :<math>\scriptstyle{\color{blue}{x=7\sdot\frac{\frac{5}{3}}{20}=\frac{\frac{35}{3}}{20}=\frac{12}{20}-\frac{4}{12\sdot20}}}</math> | |
|style="text-align:right;"|ולחשבון זה תאמ' ג"כ ה' פעמי' ז' ידות הם ל"ה ידות שהם י"ב די' פחות ד"פ וכה"ל | |style="text-align:right;"|ולחשבון זה תאמ' ג"כ ה' פעמי' ז' ידות הם ל"ה ידות שהם י"ב די' פחות ד"פ וכה"ל | ||
|- | |- | ||
| − | |'''Find the Interest''' | + | |<span style=color:green>'''Find the Interest'''</span> |
| | | | ||
|- | |- | ||
| | | | ||
| − | :{{#annot:for some dinar, some years|638|SYkq}}44) I lent 20 dinar for one year at 4 pešuṭim a month and at the end of the year I cashed some. | + | :{{#annot:for some dinar, some years|638|SYkq}}44) If a man asks you: I lent 20 dinar for one year at 4 pešuṭim a month and at the end of the year I cashed some. |
:I lent [what remained] for a second year at 4 pešuṭim a month also and cashed some. | :I lent [what remained] for a second year at 4 pešuṭim a month also and cashed some. | ||
:I lent [what remained] for a third year again at 4 pešuṭim a month and cashed some as I did in the first and the second year. | :I lent [what remained] for a third year again at 4 pešuṭim a month and cashed some as I did in the first and the second year. | ||
:Then I had no pašuṭ left. | :Then I had no pašuṭ left. | ||
:How much is the sum of all the repayments, and how much was each repayment? | :How much is the sum of all the repayments, and how much was each repayment? | ||
| − | |||
|style="text-align:right;"|מד) אם ישאלך אדם הלותי כ' די' שנה אחת לחש' דפ"ה ובסוף השנה פרעתי מהם קצת<br> | |style="text-align:right;"|מד) אם ישאלך אדם הלותי כ' די' שנה אחת לחש' דפ"ה ובסוף השנה פרעתי מהם קצת<br> | ||
הלותים שנה שניה לחשבון דפ"ה כמו כן ופרעתי מהם קצת<br> | הלותים שנה שניה לחשבון דפ"ה כמו כן ופרעתי מהם קצת<br> | ||
| Line 1,361: | Line 1,627: | ||
ולא נשאר בידי אי פשוט<br> | ולא נשאר בידי אי פשוט<br> | ||
כמה עלו כל הפרעונות וכמה כל פרעון ופרעון לבדו{{#annotend:SYkq}} | כמה עלו כל הפרעונות וכמה כל פרעון ופרעון לבדו{{#annotend:SYkq}} | ||
| + | |- | ||
| + | |colspan="2"| | ||
| + | :<math>\scriptstyle20+4-X+\left[\frac{4}{20}\sdot\left(20+4-X\right)\right]-X+\left[\frac{4}{20}\sdot\left[20+4-X+\left[\frac{4}{20}\sdot\left(20+4-X\right)\right]-X\right]\right]=X</math> | ||
|- | |- | ||
| | | | ||
| − | : | + | :The sum of all the payments is 28 dinar, 5 pešuṭim and 73 parts of one pašuṭ. |
| − | + | :<math>\scriptstyle{\color{blue}{3X=28+\frac{5+\frac{73}{91}}{12}}}</math> | |
|style="text-align:right;"|עלו כל הפרעונות כ"ח דינרי' וה' פשו' וע"ג חלקי' מצ"א בפשוט | |style="text-align:right;"|עלו כל הפרעונות כ"ח דינרי' וה' פשו' וע"ג חלקי' מצ"א בפשוט | ||
|- | |- | ||
| | | | ||
| − | :: | + | :Each payment is 9 dinar, 5 pešuṭim and 85 parts of 91. |
| − | + | :<math>\scriptstyle{\color{blue}{X=\frac{28+\frac{5+\frac{73}{91}}{12}}{3}=9+\frac{5+\frac{85}{91}}{12}}}</math> | |
|style="text-align:right;"|שיבא לכל פרעון ט' די' וה' פשוטי' ופ"ה חלקי' מצ"א | |style="text-align:right;"|שיבא לכל פרעון ט' די' וה' פשוטי' ופ"ה חלקי' מצ"א | ||
|- | |- | ||
| | | | ||
| − | :: | + | :The calculation procedure is as follows: take another number, suppose the payment in each year is 6 dinar. |
|style="text-align:right;"|ודרך עשיית החשבון הוא על זה הדרך שתתפוש החשבון אחר ותשים קצת הפרעון שבכל שנה ושנה ו' די‫' | |style="text-align:right;"|ודרך עשיית החשבון הוא על זה הדרך שתתפוש החשבון אחר ותשים קצת הפרעון שבכל שנה ושנה ו' די‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | :Take the final number, i.e. at the end of the third year 6 dinar remained. |
| − | |||
|style="text-align:right;"|ותתפוש החשבון מסופו דהיינו שבסוף שנה שלישית נשארו ו' דינרי‫' | |style="text-align:right;"|ותתפוש החשבון מסופו דהיינו שבסוף שנה שלישית נשארו ו' דינרי‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | :We want to know how much remained at the beginning of the third year, which is the end of the second year: |
| − | + | |style="text-align:right;"|ונרצה לדעת כמה נשארו בראש שנה שלישית שהוא סוף שנה שנייה | |
| − | |style="text-align:right;"|ונרצה לדעת כמה נשארו בראש שנה שלישית שהוא סוף שנה שנייה | ||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | :: | + | :Say: if 6 dinar remained at the end of the third year, subtract their sixth; the remainder is the invested money that remained at the end of the second year. |
| − | :::<math>\scriptstyle{\color{blue}{6+5=11}}</math> | + | |style="text-align:right;"|אמור אם בסוף שנה שלישית נשארו ו' דינרי' הסר מהם השתות והנשאר הוא הקרן שנשאר בסוף שנה שנייה |
| + | |- | ||
| + | | | ||
| + | :? | ||
| + | |style="text-align:right;"|כי לעולם בכל חשבון עולה בו ריוח לחשבון דפה"ה החומש מלגין שהוא שתות מלבד | ||
| + | |- | ||
| + | | | ||
| + | :So, when you subtract its sixth from it, only the invested money remains. | ||
| + | |style="text-align:right;"|ולכן כשתסיר ממנו ‫<ref>92r</ref>השתות ישאר הקרן לבד | ||
| + | |- | ||
| + | | | ||
| + | :Hence, at the beginning of the third year there were 5 dinar left. | ||
| + | :<math>\scriptstyle{\color{blue}{6-\left(\frac{1}{5+1}\sdot6\right)=6-\left(\frac{1}{6}\sdot6\right)=6-1=5}}</math> | ||
| + | |style="text-align:right;"|א"כ בראש שנה שלישית נשארו ה' די‫' | ||
| + | |- | ||
| + | | | ||
| + | :Therefore, you paid 6 dinar for the second year, so at the end of the second year there were 11. | ||
| + | :<math>\scriptstyle{\color{blue}{6+5=11}}</math> | ||
|style="text-align:right;"|ואז פרעת ו' די' עבור שנה שנייה הרי שבסוף שנה שנייה היו י"א | |style="text-align:right;"|ואז פרעת ו' די' עבור שנה שנייה הרי שבסוף שנה שנייה היו י"א | ||
|- | |- | ||
| | | | ||
| − | : | + | :Subtract their sixth, which is their profit at the second year; 9 dinar and 2 pešuṭim remain. |
| − | + | :<math>\scriptstyle{\color{blue}{11-\left(\frac{1}{6}\sdot11\right)=9+\frac{2}{12}}}</math> | |
|style="text-align:right;"|הסר מהם השתות שהוא הריוח שעלה בהם בשנה שנייה נשארו ט' די' וב"פ | |style="text-align:right;"|הסר מהם השתות שהוא הריוח שעלה בהם בשנה שנייה נשארו ט' די' וב"פ | ||
|- | |- | ||
| | | | ||
| − | : | + | :Then, you paid 6 dinar for the first year, so at the end of the first year there were 15 dinar and 2 pešuṭim. |
| − | + | :<math>\scriptstyle{\color{blue}{6+\left(9+\frac{2}{12}\right)=15+\frac{2}{12}}}</math> | |
|style="text-align:right;"|ואז פרעת ו' די' בעבור שנה ראשונה הרי שבסוף שנה ראשונה היו ט"ו די' וב"פ | |style="text-align:right;"|ואז פרעת ו' די' בעבור שנה ראשונה הרי שבסוף שנה ראשונה היו ט"ו די' וב"פ | ||
|- | |- | ||
| | | | ||
| − | : | + | :Subtract their sixth; 12 dinar, 7 pešuṭim, and 2-thirds of one pašuṭ remain. |
| − | + | :<math>\scriptstyle{\color{blue}{\left(15+\frac{2}{12}\right)-\left[\frac{1}{6}\sdot\left(15+\frac{2}{12}\right)\right]=12+\frac{7+\frac{2}{3}}{12}}}</math> | |
|style="text-align:right;"|הסר מהם השתות ישארו י"ב די' וז"פ וב' שלישי פשוט | |style="text-align:right;"|הסר מהם השתות ישארו י"ב די' וז"פ וב' שלישי פשוט | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> So, we know that if the invested money is 12 dinar, 7 pešuṭim and 2-thirds of one pašuṭ, the payment is 6 dinar each year. We want to know: if the invested money is 20 dinar, how much will be the payment? | |
| − | + | :<math>\scriptstyle{\color{blue}{\left(12+\frac{7+\frac{2}{3}}{12}\right):6=20:X}}</math> | |
|style="text-align:right;"|אם כן ידענו שאם הקרן הוא י"ב די' וז"פ וב' שלישי פשוט הפרעון הוא ו' די' בכל שנה ונרצה לידע אם הקרן הוא כ' די' כמה הוא הפרעון | |style="text-align:right;"|אם כן ידענו שאם הקרן הוא י"ב די' וז"פ וב' שלישי פשוט הפרעון הוא ו' די' בכל שנה ונרצה לידע אם הקרן הוא כ' די' כמה הוא הפרעון | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> Do as follows: if 3 equals 4, how much is 5 equal to? | |
| − | |style="text-align:right;"|עשהו בדרך זה אם ג' שוה ד' ה' כמה שוה והבן זה כי עמוק הוא וכה"ל | + | :<math>\scriptstyle{\color{blue}{3:4=5:X}}</math> |
| + | |style="text-align:right;"|עשהו בדרך זה אם ג' שוה ד' ה' כמה שוה | ||
| + | |- | ||
| + | | | ||
| + | :Understand this, for it is complicated. | ||
| + | |style="text-align:right;"|והבן זה כי עמוק הוא וכה"ל | ||
|- | |- | ||
| | | | ||
| − | *another | + | *We can also solve it another way: |
|style="text-align:right;"|גם נוכל לעשותו בדרך אחרת | |style="text-align:right;"|גם נוכל לעשותו בדרך אחרת | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\frac{\frac{1+\frac{2}{3}}{12}}{12+\frac{7+\frac{2}{3}}{12}}=\frac{1}{91}}}</math> | + | :Say: how many parts of 12 dinar, 7 pešuṭim and 2-thirds are one pašuṭ and 2-thirds? They are one part of 91. |
| + | :<math>\scriptstyle{\color{blue}{\frac{\frac{1+\frac{2}{3}}{12}}{12+\frac{7+\frac{2}{3}}{12}}=\frac{1}{91}}}</math> | ||
|style="text-align:right;"|ותאמר א"פ וב' שלשיות כמה חלק הוא מי"ב די' וז"פ וב' שלישיות הוא חלק אחד מצ"א | |style="text-align:right;"|ותאמר א"פ וב' שלשיות כמה חלק הוא מי"ב די' וז"פ וב' שלישיות הוא חלק אחד מצ"א | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\left(12+\frac{7+\frac{2}{3}}{12}\right)-\left[\frac{1}{91}\sdot\left(12+\frac{7+\frac{2}{3}}{12}\right)\right]=12+\frac{1}{2}}}</math> | + | :If you subtract it from the invested money, 12 dinar and a half remain. |
| + | :<math>\scriptstyle{\color{blue}{\left(12+\frac{7+\frac{2}{3}}{12}\right)-\left[\frac{1}{91}\sdot\left(12+\frac{7+\frac{2}{3}}{12}\right)\right]=12+\frac{1}{2}}}</math> | ||
|style="text-align:right;"|אם תסירהו מן הקרן ישארו י"ב די' וחצי | |style="text-align:right;"|אם תסירהו מן הקרן ישארו י"ב די' וחצי | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\left(12+\frac{1}{2}\right)-\left[\frac{1}{5}\sdot\left(12+\frac{1}{2}\right)\right]=10}}</math> | + | :Subtract from the remainder its fifth also; 10 dinar remain. |
| + | :<math>\scriptstyle{\color{blue}{\left(12+\frac{1}{2}\right)-\left[\frac{1}{5}\sdot\left(12+\frac{1}{2}\right)\right]=10}}</math> | ||
|style="text-align:right;"|תסיר ממנו עוד מן הנשאר החומש ישארו י' די‫' | |style="text-align:right;"|תסיר ממנו עוד מן הנשאר החומש ישארו י' די‫' | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{2\sdot10=20}}</math> | + | :Double it; the result is 20 dinar. |
| + | :<math>\scriptstyle{\color{blue}{2\sdot10=20}}</math> | ||
|style="text-align:right;"|כפלהו יעלה כ' די‫' | |style="text-align:right;"|כפלהו יעלה כ' די‫' | ||
|- | |- | ||
| | | | ||
| − | ::each payment | + | :Do the same with the payment: subtract one part of 91 from 6 dinar. Then, subtract from the remainder its fifth and double the remainder; you receive 9 dinar, 5 pešuṭim and 85 parts of 91 and so is each payment. |
| − | + | :<math>\scriptstyle{\color{blue}{2\sdot\left[\left[6-\left(\frac{1}{91}\sdot6\right)\right]-\left[\frac{1}{5}\sdot\left[6-\left(\frac{1}{91}\sdot6\right)\right]\right]\right]=9+\frac{5+\frac{85}{91}}{12}}}</math> | |
| − | |||
|style="text-align:right;"|וכן תעשה בפרעון תסיר מו' די' חלק אחד מצ"א<br> | |style="text-align:right;"|וכן תעשה בפרעון תסיר מו' די' חלק אחד מצ"א<br> | ||
אחרי כן תסיר מן הנשאר החומש ותכפול המותר<br> | אחרי כן תסיר מן הנשאר החומש ותכפול המותר<br> | ||
יעלה בידך ט' די' וה"פ ופ"ה חלקי' מצ"א וכן יבא לכל פרעון ופרעון וכה"ל | יעלה בידך ט' די' וה"פ ופ"ה חלקי' מצ"א וכן יבא לכל פרעון ופרעון וכה"ל | ||
|- | |- | ||
| − | |'''Pricing Problem - Find the Price''' | + | |<span style=color:green>'''Pricing Problem - Find the Price'''</span> |
| | | | ||
|- | |- | ||
| | | | ||
| − | :{{#annot:fruit|629|oVVn}}45) You bought some fruit in trade at so and so liṭra for one kikkar and you want to know how much one liṭra is worth | + | :{{#annot:fruit|629|oVVn}}45) You bought some fruit in trade at so and so liṭra for one kikkar and you want to know how much one liṭra is worth in a short way. |
:<math>\scriptstyle\frac{20\sdot a}{100}=\frac{X}{1}</math> | :<math>\scriptstyle\frac{20\sdot a}{100}=\frac{X}{1}</math> | ||
| − | |style="text-align:right;"|מה | + | |style="text-align:right;"|מה <big>פרי</big> שקנית תגרות בכל כך ליט' הככר ותרצה לידע כמה יבא הליט' התגרות בדרך קצרה{{#annotend:oVVn}} |
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle X=\frac{a}{5}</math> | + | :Take a fifth of the amount of money in cash and subtract 4-fifths. For every liṭra you have left take one dinar and that is the value in cash of the liṭra in the trade. |
| + | :<math>\scriptstyle X=\frac{a}{5}</math> | ||
|style="text-align:right;"|תפוש החומש מסכום החשבון המעות וד' חומשי' תשליך ומכל ליט' שנשאר בידך קח די' וכל כך מעות יבא הליט' מן התגרות | |style="text-align:right;"|תפוש החומש מסכום החשבון המעות וד' חומשי' תשליך ומכל ליט' שנשאר בידך קח די' וכל כך מעות יבא הליט' מן התגרות | ||
|- | |- | ||
| | | | ||
| − | + | *{{#annot:pepper|629|sShl}}Example: I bought 100 liṭra of pepper for 40 liṭra and you want to know how much one liṭra is worth. | |
| − | + | :<math>\scriptstyle\frac{20\sdot40}{100}=\frac{X}{1}</math> | |
|style="text-align:right;"|המשל בזה הרי שקניתי ק' ליט' מפלפל לחשבון מ' ליט' ותרצה לידע כמה יבא הליט‫'{{#annotend:sShl}} | |style="text-align:right;"|המשל בזה הרי שקניתי ק' ליט' מפלפל לחשבון מ' ליט' ותרצה לידע כמה יבא הליט‫'{{#annotend:sShl}} | ||
|- | |- | ||
| | | | ||
| − | : | + | :Take the fifth that are 8 liṭra. For every liṭra take one dinar; the result is 8 dinar. So, one liṭra of pepper is worth 8 dinar. |
| − | |style="text-align:right;"|תפוש החומש שהם ח' ליט' ומכל | + | :<math>\scriptstyle{\color{blue}{x=\frac{40}{5}=8}}</math> |
| + | |style="text-align:right;"|תפוש החומש שהם ח' ליט' ומכל הליט' תקח די' הרי שיבא ח' די' הרי שיבא הליט' מן הפלפל ח' די' וה"ל | ||
|- | |- | ||
| | | | ||
| − | : | + | :Since, one kikkar in trade is worth one liṭra in cash, every liṭra is worth 2 pešuṭim and 2-fifths [in cash]. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{\frac{12}{5}=2+\frac{2}{5}}}</math> |
| + | |style="text-align:right;"|אחר שבכל ליט' ממעות שהוציא בככר אחד מן התגרות יגיע לכל ליט' ב"פ וב' חמשי‫' | ||
|- | |- | ||
| | | | ||
| − | + | *If you bought one kikkar in trade for 40 liṭra and you want to know how much one liṭra is worth. | |
|style="text-align:right;"|והרי שקנית הככר מן התגרות במ' ליט' ותרצה לידע כמה יבא הליט‫' | |style="text-align:right;"|והרי שקנית הככר מן התגרות במ' ליט' ותרצה לידע כמה יבא הליט‫' | ||
|- | |- | ||
| | | | ||
| − | ::: | + | :Say: 2 times 40 pešuṭim and 2 times 40-fifths of one pašuṭ are 8 dinar. |
| + | :<math>\scriptstyle{\color{blue}{\left(2+\frac{2}{5}\right)\sdot\frac{40}{12}=\left(2\sdot\frac{40}{12}\right)+\left(2\sdot\frac{\frac{40}{5}}{12}\right)=8}}</math> | ||
|style="text-align:right;"|תאמר ב"פ מ"פ וב' פעמי' מ' חומשי פשוט הם ח' די' וכה"ל | |style="text-align:right;"|תאמר ב"פ מ"פ וב' פעמי' מ' חומשי פשוט הם ח' די' וכה"ל | ||
|- | |- | ||
| − | |'''Find the Earned Interest''' | + | |<span style=color:green>'''Find the Earned Interest'''</span> |
| | | | ||
|- | |- | ||
| | | | ||
| − | :{{#annot:for some liṭra a day|638|AHu7}}46) How much will be the profit of 100 liṭra for one day at 8 liṭra a year? | + | :{{#annot:for some liṭra a day|638|AHu7}}46) If a man asks you: How much will be the profit of 100 liṭra for one day at 8 liṭra a year? |
:<math>\scriptstyle\frac{\left(20\sdot12\right)\sdot8}{360\sdot100}=\frac{X}{1\sdot100}</math> | :<math>\scriptstyle\frac{\left(20\sdot12\right)\sdot8}{360\sdot100}=\frac{X}{1\sdot100}</math> | ||
| − | |style="text-align:right;"|מו | + | |style="text-align:right;"|מו <big>אם</big> ישאלך אדם כמה יעלה הריוח מק' ליט' א' יום לחשבון ח' ליט' השנה{{#annotend:AHu7}} |
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> Say: 2 times 8 is 16. Take the third in peruṭot, which are 5 pešuṭim and a third. | |
| + | :<math>\scriptstyle{\color{blue}{x=\frac{2\sdot8}{3}=\frac{16}{3}=5+\frac{1}{3}}}</math> | ||
|style="text-align:right;"|תאמר ב' פעמי' ח' הם י"ו תפוש השליש בפרוטות שהם ה"פ ושליש וכן יבא וכה"ל | |style="text-align:right;"|תאמר ב' פעמי' ח' הם י"ו תפוש השליש בפרוטות שהם ה"פ ושליש וכן יבא וכה"ל | ||
|- | |- | ||
| | | | ||
| − | == Problems of Various Types == | + | == <span style=color:green>Problems of Various Types</span> == |
| | | | ||
|- | |- | ||
| − | | | + | | |
| + | === <span style=color:green>Rent Problem</span> === | ||
| + | |||
| | | | ||
|- | |- | ||
| Line 1,503: | Line 1,799: | ||
:{{#annot:a day|615|pFZr}}47) If you rent a house at so and so liṭra a year and you want to know how much [should be paid] a day | :{{#annot:a day|615|pFZr}}47) If you rent a house at so and so liṭra a year and you want to know how much [should be paid] a day | ||
:<math>\scriptstyle\frac{\left(20\sdot12\right)\sdot a}{360}=\frac{X}{1}</math> | :<math>\scriptstyle\frac{\left(20\sdot12\right)\sdot a}{360}=\frac{X}{1}</math> | ||
| − | |style=" | + | |style="text-align:right;"|מז <big>אם</big> תשכיר בית אחד לחשבון כך וכך ליט' השנה ותרצה לידע כמה יבא היום{{#annotend:pFZr}} |
|- | |- | ||
| | | | ||
| − | :: | + | :Do as follows: every liṭra spent on the house a year gives 2-thirds of a pašuṭ per day. |
| − | + | :<math>\scriptstyle X=a\sdot\frac{2}{3}</math> | |
|style="text-align:right;"|עשה כן מכל ליט' שתוציא בבית השנה יבא ליום ב' שלישי פשוט | |style="text-align:right;"|עשה כן מכל ליט' שתוציא בבית השנה יבא ליום ב' שלישי פשוט | ||
|- | |- | ||
| | | | ||
| − | + | *{{#annot:a day|615|kyHM}}You rent a house at 20 liṭra a year. | |
| − | + | :<math>\scriptstyle\frac{\left(20\sdot12\right)\sdot20}{360}=\frac{X}{1}</math> | |
|style="text-align:right;"|והרי שהשכרת בית אחד לחשבון כ' ליט' השנה{{#annotend:kyHM}} | |style="text-align:right;"|והרי שהשכרת בית אחד לחשבון כ' ליט' השנה{{#annotend:kyHM}} | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{x=20\sdot\frac{2}{3}=\frac{40}{3}=13+\frac{1}{3}}}</math> | + | :Say: 20 times 2-thirds are 40, which are 13 pešuṭim and one third. |
| + | :<math>\scriptstyle{\color{blue}{x=20\sdot\frac{2}{3}=\frac{40}{3}=13+\frac{1}{3}}}</math> | ||
|style="text-align:right;"|תאמ' כ' פעמי' ב שלשיים הם מ' שהם י"ג פשו' ושלי' וכן יבא לזו | |style="text-align:right;"|תאמ' כ' פעמי' ב שלשיים הם מ' שהם י"ג פשו' ושלי' וכן יבא לזו | ||
|- | |- | ||
| − | | | + | | |
| + | |||
| + | === <span style=color:green>Exchange Problems</span> === | ||
| + | |||
| | | | ||
|- | |- | ||
| | | | ||
| − | :{{#annot:two currencies|632|qKNX}}48) 22 of Pisa are worth 25 of Bologna. | + | :{{#annot:two currencies|632|qKNX}}48) If a man asks you: 22 of Pisa are worth 25 of Bologna. |
:What is the exchange of the liṭra of Bologna to a liṭra of Pisa? | :What is the exchange of the liṭra of Bologna to a liṭra of Pisa? | ||
:<math>\scriptstyle\frac{22}{25}=\frac{1}{X}</math> | :<math>\scriptstyle\frac{22}{25}=\frac{1}{X}</math> | ||
| − | |style="text-align:right;"|מח | + | |style="text-align:right;"|מח <big>אם</big> ישאלך אדם הכ"ב פיסני שוים כ"ה בולייניני' כמה יתן חלוף הליט' מן הבולונייני' לליט' מן הפיסני‫'{{#annotend:qKNX}} |
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> Multiply 25 times 1 liṭra of Bologna; they are 25 liṭra of Bologna. Divide them by 22; the result is 20 dinar, 8 pešuṭim and 8 parts of 11 of one pašuṭ, and this is the exchange of a liṭra of Bologna to a liṭra of Pisa. | |
| − | + | :<math>\scriptstyle{\color{blue}{x=\frac{25\sdot1}{22}=\frac{25}{22}=\frac{20}{20}+\frac{\frac{8}{11}}{20}}}</math> | |
|style="text-align:right;"|תרבה כ"ה פעמי' א' ליט' מבולנייני' יהיו כ"ה ליט' מבולנייני‫'<br> | |style="text-align:right;"|תרבה כ"ה פעמי' א' ליט' מבולנייני' יהיו כ"ה ליט' מבולנייני‫'<br> | ||
| − | חלקם על כ"ב יבא כ' די' וח"פ | + | חלקם על כ"ב יבא כ' די' וח"פ וח' חלקי' מי"א בפשוט<br> |
וכן יתן חלוף הליט' מן הבוליי' לליט' מן הפיסני‫' | וכן יתן חלוף הליט' מן הבוליי' לליט' מן הפיסני‫' | ||
|- | |- | ||
| − | | | + | | |
| + | |||
| + | === <span style=color:green>Proportions of Fractions</span> === | ||
| + | |||
| | | | ||
|- | |- | ||
| Line 1,541: | Line 1,844: | ||
:{{#annot:⅓÷¼=⅕÷X|567|UdQe}}49) If one third equals a quarter, how much is one fifth equal? | :{{#annot:⅓÷¼=⅕÷X|567|UdQe}}49) If one third equals a quarter, how much is one fifth equal? | ||
:<math>\scriptstyle\frac{\frac{1}{3}}{\frac{1}{4}}=\frac{\frac{1}{5}}{X}</math> | :<math>\scriptstyle\frac{\frac{1}{3}}{\frac{1}{4}}=\frac{\frac{1}{5}}{X}</math> | ||
| − | |style="text-align:right;"|מט | + | |style="text-align:right;"|מט <big>אם</big> שליש שוה רביע חומש כמה שוה{{#annotend:UdQe}} |
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''False Position = commen denominator:'''</span> First, say: where are a third, a quarter, and a fifth to be found? Say: 3 times 4 is 12; 5 times 12 is 60. | |
| − | + | :<math>\scriptstyle{\color{blue}{3\sdot4\sdot5=12\sdot5=60}}</math> | |
|style="text-align:right;"|אמור תחלה אנה ימצאו שליש ורביע וחומש<br> | |style="text-align:right;"|אמור תחלה אנה ימצאו שליש ורביע וחומש<br> | ||
אמור ג' פעמי' ד' י"ב<br> | אמור ג' פעמי' ד' י"ב<br> | ||
| Line 1,551: | Line 1,854: | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\frac{1}{3}\sdot60=20}}</math> | + | :The third is 20. |
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{3}\sdot60=20}}</math> | ||
|style="text-align:right;"|השליש הוא כ‫' | |style="text-align:right;"|השליש הוא כ‫' | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\frac{1}{4}\sdot60=15}}</math> | + | :The quarter is 15. |
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{4}\sdot60=15}}</math> | ||
|style="text-align:right;"|הרביע הוא ט"ו | |style="text-align:right;"|הרביע הוא ט"ו | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\frac{1}{5}\sdot60=12}}</math> | + | :The fifth is 12. |
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{5}\sdot60=12}}</math> | ||
|style="text-align:right;"|החומש הוא י"ב | |style="text-align:right;"|החומש הוא י"ב | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> Say: if 20 equals 15, how much is 12 equal to? | |
| + | :<math>\scriptstyle{\color{blue}{20:15=12:\left(60\sdot X\right)}}</math> | ||
|style="text-align:right;"|ואמור אם כ' שוה ט"ו י"ב כמה שוה | |style="text-align:right;"|ואמור אם כ' שוה ט"ו י"ב כמה שוה | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{X=\frac{\frac{12\sdot15}{20}}{60}=\frac{\frac{180}{20}}{60}=\frac{9}{60}=\frac{3}{20}}}</math> | + | :Say: 12 times 15 is 180. Divide it into 20 parts; the result is 9, which are 9 parts of 60, which are 3 parts of 20. |
| + | :<math>\scriptstyle{\color{blue}{X=\frac{\frac{12\sdot15}{20}}{60}=\frac{\frac{180}{20}}{60}=\frac{9}{60}=\frac{3}{20}}}</math> | ||
|style="text-align:right;"|אמור י"ב פעמי' ט"ו הם ק"פ<br> | |style="text-align:right;"|אמור י"ב פעמי' ט"ו הם ק"פ<br> | ||
חלקם בכ' חלקי' הרי שיבא ט' שהם ט' חלקי' מס‫'<br> | חלקם בכ' חלקי' הרי שיבא ט' שהם ט' חלקי' מס‫'<br> | ||
שהם ג' חלקי' מכ' וכה"ל | שהם ג' חלקי' מכ' וכה"ל | ||
| − | |||
| − | |||
|- | |- | ||
| − | + | | | |
| + | |||
| + | === <span style=color:green>Find a Quantity Problem - Whole from Parts – Tree</span> === | ||
| + | |||
| | | | ||
|- | |- | ||
| Line 1,580: | Line 1,889: | ||
:{{#annot:tree|650|tA4t}}50) If one asks you: I have a tree - its half and its third are in the water; a third and a quarter of what is left are planted in the soil; and three spans remain. | :{{#annot:tree|650|tA4t}}50) If one asks you: I have a tree - its half and its third are in the water; a third and a quarter of what is left are planted in the soil; and three spans remain. | ||
:How much is the length of the tree? | :How much is the length of the tree? | ||
| − | + | |style="text-align:right;"|‫<ref>92v</ref>נ <big>אם</big> ישאלך אדם יש לי אי אילן שהחצי והשליש ממנו הוא עומד במים תחת הארץ<br> | |
| − | |style=" | ||
והשליש והרבע מן הנשאר הוא מכוסה תקוע בארץ<br> | והשליש והרבע מן הנשאר הוא מכוסה תקוע בארץ<br> | ||
ונשאר ממנו מן האילן ג' זרתות<br> | ונשאר ממנו מן האילן ג' זרתות<br> | ||
אשאלך כמה גבהות כל האילן{{#annotend:tA4t}} | אשאלך כמה גבהות כל האילן{{#annotend:tA4t}} | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | :<math>\scriptstyle\left(\frac{1}{2}X+\frac{1}{3}X\right)+\left[\left(\frac{1}{3}+\frac{1}{4}\right)\sdot\left[X-\left(\frac{1}{2}X+\frac{1}{3}X\right)\right]\right]+3=X</math> | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''False Position = common denominator:'''</span> Look for the denominator, which is 72, by multiplying 3 by 2, which is 6; 3 by 6 is 18; 4 by 18 is 72. | |
| − | + | :<math>\scriptstyle{\color{blue}{3\sdot2\sdot3\sdot4=6\sdot3\sdot4=18\sdot4=72}}</math> | |
|style="text-align:right;"|בקש המורה והוא ע"ב בכפלך ג' על ב' והם ו' וג' פעמי' ו' הם י"ח וד' פעמי' י"ח הם ע"ב | |style="text-align:right;"|בקש המורה והוא ע"ב בכפלך ג' על ב' והם ו' וג' פעמי' ו' הם י"ח וד' פעמי' י"ח הם ע"ב | ||
|- | |- | ||
| | | | ||
| − | + | :So, you find in 72 a half, a third, a third, and a quarter. | |
|style="text-align:right;"|ובע"ב תמצא חצי ושליש ושליש ורבע | |style="text-align:right;"|ובע"ב תמצא חצי ושליש ושליש ורבע | ||
|- | |- | ||
| | | | ||
| − | + | :Extract a third and a half from 72; it is 60 and 12 remains. | |
| − | + | :<math>\scriptstyle{\color{blue}{72-\left[\left(\frac{1}{2}\sdot72\right)+\left(\frac{1}{3}\sdot72\right)\right]=72-60=12}}</math> | |
|style="text-align:right;"|והוצא שליש וחצי מע"ב והם ס' וישארו י"ב | |style="text-align:right;"|והוצא שליש וחצי מע"ב והם ס' וישארו י"ב | ||
|- | |- | ||
| | | | ||
| − | + | :For the part that is in the ground, extract a third and a quarter of 12; it is 7. | |
| − | + | :<math>\scriptstyle{\color{blue}{\left(\frac{1}{3}\sdot12\right)+\left(\frac{1}{4}\sdot12\right)=7}}</math> | |
|style="text-align:right;"|א"כ בעבור החלק האחד שהוא בארץ הוצא השליש והרבע מי"ב והם ז‫' | |style="text-align:right;"|א"כ בעבור החלק האחד שהוא בארץ הוצא השליש והרבע מי"ב והם ז‫' | ||
|- | |- | ||
| | | | ||
| − | + | :Now, sum 7 with 60; it is 67 and 5 remains. | |
| − | + | :<math>\scriptstyle{\color{blue}{72-\left(60+7\right)=72-67=5}}</math> | |
|style="text-align:right;"|ועתה {{#annot:term|178,1190|7LIm}}אסוף{{#annotend:7LIm}} ז' עם ס' והם ס"ז וישארו ה‫' | |style="text-align:right;"|ועתה {{#annot:term|178,1190|7LIm}}אסוף{{#annotend:7LIm}} ז' עם ס' והם ס"ז וישארו ה‫' | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> Do [as follows:] if 5 is 3, how much is 72? | |
| − | + | :<math>\scriptstyle{\color{blue}{5:3=72:X}}</math> | |
|style="text-align:right;"|אם כן עשה אם ה' היו ג' ע"ב כמה היו | |style="text-align:right;"|אם כן עשה אם ה' היו ג' ע"ב כמה היו | ||
|- | |- | ||
| | | | ||
| − | + | :72 times 3 is 216. | |
|style="text-align:right;"|ע"ב פעמי' ג' הם רי"ו | |style="text-align:right;"|ע"ב פעמי' ג' הם רי"ו | ||
|- | |- | ||
| | | | ||
| − | + | :Divide it by 5, the result is 43 and a fifth. | |
|style="text-align:right;"|חלקם בה' בא מ"ג וחומש | |style="text-align:right;"|חלקם בה' בא מ"ג וחומש | ||
|- | |- | ||
| | | | ||
| − | :Hence, the length of the whole tree is | + | :Hence, the length of the whole tree is 43 spans and a fifth. |
:<math>\scriptstyle{\color{blue}{x=\frac{72\sdot3}{5}=\frac{216}{5}=43+\frac{1}{5}}}</math> | :<math>\scriptstyle{\color{blue}{x=\frac{72\sdot3}{5}=\frac{216}{5}=43+\frac{1}{5}}}</math> | ||
| − | |style="text-align:right;"|א"כ אורך כל האילן היה מ"ג זרתות וחומש | + | |style="text-align:right;"|א"כ אורך כל האילן היה מ"ג זרתות וחומש וכה"ל |
|- | |- | ||
| | | | ||
| − | + | ||
| − | + | === <span style=color:green>Motion Problem - To and From - an Ant Climbing a Tower</span> === | |
| − | + | ||
| − | |||
| − | |||
| | | | ||
|- | |- | ||
| | | | ||
| − | :{{#annot:ant climbing | + | :{{#annot:ant climbing|659|nnul}}51) There is a tower in front of you that is 20 cubits high. |
:An ant wants to climb up. | :An ant wants to climb up. | ||
:Every day it climbs up one third of a cubit and every night it goes down a quarter [of a cubit]. | :Every day it climbs up one third of a cubit and every night it goes down a quarter [of a cubit]. | ||
:In how many days will it reach the top? | :In how many days will it reach the top? | ||
:<math>\scriptstyle\frac{1}{3}X-\frac{1}{4}X=20</math> | :<math>\scriptstyle\frac{1}{3}X-\frac{1}{4}X=20</math> | ||
| − | |style="text-align:right;"|נא | + | |style="text-align:right;"|נא <big>הרי</big> שיש לפניך מגדל גבוה כ' אמה<br> |
ונמלה אחת רוצה לעלות למעלה<br> | ונמלה אחת רוצה לעלות למעלה<br> | ||
ובכל יום עולה שליש אמה ובכל לילה יורדת רביע<br> | ובכל יום עולה שליש אמה ובכל לילה יורדת רביע<br> | ||
| Line 1,648: | Line 1,957: | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\frac{1}{3}-\frac{1}{4}=\frac{1}{12}}}</math> | + | :First, say: by how much is the third greater than the quarter? By one part of 12. |
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{3}-\frac{1}{4}=\frac{1}{12}}}</math> | ||
|style="text-align:right;"|אמור תחלה כמה יותר השליש מן הרביע א' חלק מי"ב | |style="text-align:right;"|אמור תחלה כמה יותר השליש מן הרביע א' חלק מי"ב | ||
|- | |- | ||
| | | | ||
| − | : | + | :We find that each day it [moves upwards] by one part of 12 of a cubit. |
|style="text-align:right;"|נמצא שבכל יום יש לה יתרון א' חלק מי"ב מן האמה | |style="text-align:right;"|נמצא שבכל יום יש לה יתרון א' חלק מי"ב מן האמה | ||
|- | |- | ||
| | | | ||
| − | : | + | :So, it climbs up one cubit in 12 days. |
|style="text-align:right;"|הרי שבי"ב ימים היא עולה אמה אחת | |style="text-align:right;"|הרי שבי"ב ימים היא עולה אמה אחת | ||
|- | |- | ||
| | | | ||
| − | ::it will reach the top in <math>\scriptstyle{\color{blue}{x=12\sdot20=240}}</math> | + | :Say: 12 times 20, for the tower is 20 [cubits] high; it is 240. Therefore, it will reach [the top of] the tower in 240 days. |
| + | :<math>\scriptstyle{\color{blue}{x=12\sdot20=240}}</math> | ||
|style="text-align:right;"|אמור י"ב פעמים כ' בעבור המגדל שגובהו כ' הרי ר"מ<br> | |style="text-align:right;"|אמור י"ב פעמים כ' בעבור המגדל שגובהו כ' הרי ר"מ<br> | ||
הרי שבר"מ ימים תעלה על המגדל | הרי שבר"מ ימים תעלה על המגדל | ||
|- | |- | ||
| − | | | + | | |
| + | |||
| + | === <span style=color:green>Find a Quantity Problem - First from Last Problem – Money</span> === | ||
| + | |||
| | | | ||
|- | |- | ||
| Line 1,671: | Line 1,985: | ||
:You take a third, a quarter, and a fifth of it and their sum is nine. | :You take a third, a quarter, and a fifth of it and their sum is nine. | ||
:How much remains? | :How much remains? | ||
| − | + | :<math>\scriptstyle X-\left(\frac{1}{3}X+\frac{1}{4}X+\frac{1}{5}X\right)=X-9</math> | |
| − | |style="text-align:right;"|נב | + | |style="text-align:right;"|נב <big>הרי</big> שיש לך מעות בכיס<br> |
והוצאת מהם השליש והרביע והחומש והם ט‫'<br> | והוצאת מהם השליש והרביע והחומש והם ט‫'<br> | ||
כמה יהיו הנשארים{{#annotend:ptLd}} | כמה יהיו הנשארים{{#annotend:ptLd}} | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''False Position = common denominator:'''</span> First, say: where are a third, a quarter, and a fifth? In 60. | |
|style="text-align:right;"|אמור תחלה אנה ימצאו שליש ורביע וחומש בס‫' | |style="text-align:right;"|אמור תחלה אנה ימצאו שליש ורביע וחומש בס‫' | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\frac{1}{3}\sdot60=20}}</math> | + | :The third is 20. |
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{3}\sdot60=20}}</math> | ||
|style="text-align:right;"|השליש הוא כ‫' | |style="text-align:right;"|השליש הוא כ‫' | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\frac{1}{4}\sdot60=15}}</math> | + | :The quarter is 15. |
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{4}\sdot60=15}}</math> | ||
|style="text-align:right;"|והרביע ט"ו | |style="text-align:right;"|והרביע ט"ו | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\frac{1}{5}\sdot60=12}}</math> | + | :The fifth is 12. |
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{5}\sdot60=12}}</math> | ||
|style="text-align:right;"|והחומש י"ב | |style="text-align:right;"|והחומש י"ב | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\frac{1}{3}+\frac{1}{4}+\frac{1}{5}=\frac{20+15+12}{60}=\frac{47}{60}}}</math> | + | :Their sum is 47 parts of 60. |
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{3}+\frac{1}{4}+\frac{1}{5}=\frac{20+15+12}{60}=\frac{47}{60}}}</math> | ||
|style="text-align:right;"|וכללם מ"ז חלקי' מס‫' | |style="text-align:right;"|וכללם מ"ז חלקי' מס‫' | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\frac{60}{60}-\frac{47}{60}=\frac{13}{60}}}</math> | + | :We find that the remainder is 13 parts of 60. |
| + | :<math>\scriptstyle{\color{blue}{\frac{60}{60}-\frac{47}{60}=\frac{13}{60}}}</math> | ||
|style="text-align:right;"|נמצא שהנשארי' הם י"ג חלקי' מס‫' | |style="text-align:right;"|נמצא שהנשארי' הם י"ג חלקי' מס‫' | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> Say: if 47 parts of 60 are equal to 9 pešuṭim, how much are 13 parts of 60? | |
| + | :<math>\scriptstyle{\color{blue}{\frac{47}{60}:9=\frac{13}{60}:a}}</math> | ||
|style="text-align:right;"|ואמור אם מ"ז חלקי' מס' שוים ט' פשוט י"ג חלקי' מס' כמה יבואו | |style="text-align:right;"|ואמור אם מ"ז חלקי' מס' שוים ט' פשוט י"ג חלקי' מס' כמה יבואו | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{a=\frac{13\sdot9}{47}=\frac{117}{47}=2+\frac{23}{47}}}</math> | + | :Say: 13 times 9 is 117. Divide it by 47; the result is 2 and 23 parts of 47. |
| + | :<math>\scriptstyle{\color{blue}{a=\frac{13\sdot9}{47}=\frac{117}{47}=2+\frac{23}{47}}}</math> | ||
|style="text-align:right;"|אמור י"ג פעמי' ט' הם קי"ז חלקם במ"ז בא ב' וכ"ג חלקי' ממ"ז | |style="text-align:right;"|אמור י"ג פעמי' ט' הם קי"ז חלקם במ"ז בא ב' וכ"ג חלקי' ממ"ז | ||
|- | |- | ||
| | | | ||
| − | : | + | :We find that the money that was in the purse is 11 and 23 parts of 47. |
| + | :<math>\scriptstyle{\color{blue}{X=11+\frac{23}{47}}}</math> | ||
|style="text-align:right;"|נמצא שהיו בתוך הכיס י"א וכ"ג חלקי' ממ"ז | |style="text-align:right;"|נמצא שהיו בתוך הכיס י"א וכ"ג חלקי' ממ"ז | ||
|- | |- | ||
| | | | ||
| − | : | + | :9 were taken out and the remainder is 2 and 23 parts of 47. |
| − | |style="text-align:right;"|ומה שהוציא היו ט' ומה שנשאר היו ב' וכ"ג חלקי' ממ"ז | + | :<math>\scriptstyle{\color{blue}{\left(11+\frac{23}{47}\right)-9=2+\frac{23}{47}}}</math> |
| + | |style="text-align:right;"|ומה שהוציא היו ט' ומה שנשאר היו ב' וכ"ג חלקי' ממ"ז וכה"ל | ||
|- | |- | ||
| − | |||
| | | | ||
| − | + | :{{#annot:money in purse|651|Rjq6}}53) If a man asks you: I have some money in your purse. | |
| − | + | :I subtract a tenth from it and ten remain. | |
| − | :{{#annot:money in purse|651|Rjq6}}53) | + | :How much was the money at first? |
| − | : | ||
| − | :How much was the money at first? | ||
:<math>\scriptstyle X-\left(\frac{1}{10}X\right)=10</math> | :<math>\scriptstyle X-\left(\frac{1}{10}X\right)=10</math> | ||
| − | |style="text-align:right;"|נג | + | |style="text-align:right;"|נג <big>אם</big> ישאלך אדם היו לי מעות בכיס<br> |
והוצאתי מהם העשירית ונשאר י‫'<br> | והוצאתי מהם העשירית ונשאר י‫'<br> | ||
כמה היו בתחלה{{#annotend:Rjq6}} | כמה היו בתחלה{{#annotend:Rjq6}} | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''False Position:'''</span> Take one number and say: when you take from 10 its tenth, 9 remains. | |
| − | + | :<math>\scriptstyle{\color{blue}{10-\left(\frac{1}{10}\sdot10\right)=9}}</math> | |
|style="text-align:right;"|תפוש חשבון אחד ואמור מי' כשתקח ממנו העשירית ישארו ט‫' | |style="text-align:right;"|תפוש חשבון אחד ואמור מי' כשתקח ממנו העשירית ישארו ט‫' | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> So, this is the way: if 9 is 10, how much is 1? | |
| + | :<math>\scriptstyle{\color{blue}{9:10=1:a}}</math> | ||
|style="text-align:right;"|ואם כן הדרך היא כך אם ט' היו י' א' כמה היא | |style="text-align:right;"|ואם כן הדרך היא כך אם ט' היו י' א' כמה היא | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{a=\frac{1\sdot10}{9}=\frac{10}{9}=1+\frac{1}{9}}}</math> | + | :Multiply 1 by 10; it is 10. Divide it by 9; it is 1 and a ninth. |
| + | :<math>\scriptstyle{\color{blue}{a=\frac{1\sdot10}{9}=\frac{10}{9}=1+\frac{1}{9}}}</math> | ||
|style="text-align:right;"|כפול א' על י' הם י‫'<br> | |style="text-align:right;"|כפול א' על י' הם י‫'<br> | ||
חלקם על ט' יהיו א' ותשיעי‫' | חלקם על ט' יהיו א' ותשיעי‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | :Add it to 10; it is 11 and a ninth [= the money that was in the purse]. |
| + | :<math>\scriptstyle{\color{blue}{X=10+\left(1+\frac{1}{9}\right)=11+\frac{1}{9}}}</math> | ||
|style="text-align:right;"|והוסיפם על הי' יהיו י"א ותשיעי‫' | |style="text-align:right;"|והוסיפם על הי' יהיו י"א ותשיעי‫' | ||
|- | |- | ||
| | | | ||
| − | :Check: <math>\scriptstyle{\color{blue}{11+\frac{1}{9}-\left[\frac{1}{10}\sdot\left(11+\frac{1}{9}\right)\right]=10}}</math> | + | :<span style=color:green>'''Check:'''</span> Subtract its tenth from it; 10 integers remain. |
| + | :<math>\scriptstyle{\color{blue}{11+\frac{1}{9}-\left[\frac{1}{10}\sdot\left(11+\frac{1}{9}\right)\right]=10}}</math> | ||
|style="text-align:right;"|הוצא מהם העשירית ישארו י' שלמי' וכה"ל | |style="text-align:right;"|הוצא מהם העשירית ישארו י' שלמי' וכה"ל | ||
|- | |- | ||
| | | | ||
| − | *If ten and a sixth remain | + | *If it is said that ten and a sixth remain: |
:<math>\scriptstyle X-\left(\frac{1}{10}X\right)=10+\frac{1}{6}</math> | :<math>\scriptstyle X-\left(\frac{1}{10}X\right)=10+\frac{1}{6}</math> | ||
| − | |style="text-align:right;"|ואם יאמר | + | |style="text-align:right;"|ואם יאמר כי נשארו י' ושתות |
| + | |- | ||
| + | | | ||
| + | :Do as follows: | ||
| + | |style="text-align:right;"|עשה כן | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> Since you know that in the other case it is one and a ninth, say: if one is one and a ninth, how much is a sixth? | |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{1:\left(1+\frac{1}{9}\right)=\frac{1}{6}:a}}</math> |
| + | |style="text-align:right;"|אחר שידעת כי האחר היא אחד ותשיעי‫'<br> | ||
תאמ' אם אחת היא אחת ותשיעי' השתות כמה היא | תאמ' אם אחת היא אחת ותשיעי' השתות כמה היא | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{a=\frac{1}{6}\sdot\left(1+\frac{1}{9}\right)}}</math> | + | :Take the sixth of 1 and a ninth. |
| + | :<math>\scriptstyle{\color{blue}{a=\frac{1}{6}\sdot\left(1+\frac{1}{9}\right)}}</math> | ||
|style="text-align:right;"|תפוש השתות מא' ותשיעי‫' | |style="text-align:right;"|תפוש השתות מא' ותשיעי‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | :Add it to 11 and a ninth and so it [the money that was in the purse]. |
| + | :<math>\scriptstyle{\color{blue}{X=11+\frac{1}{9}+\frac{1}{6}\sdot\left(1+\frac{1}{9}\right)}}</math> | ||
|style="text-align:right;"|והוסיפם על הי"א ותשיעי' וכן יצא | |style="text-align:right;"|והוסיפם על הי"א ותשיעי' וכן יצא | ||
|- | |- | ||
| − | | | + | | |
| + | |||
| + | === <span style=color:green>Divide a Quantity – Money</span> === | ||
| + | |||
| | | | ||
|- | |- | ||
| Line 1,774: | Line 2,108: | ||
:{{#annot:money|645|q2Sp}}54) If you want to divide 5 pešuṭim to a third and a quarter without a remainder | :{{#annot:money|645|q2Sp}}54) If you want to divide 5 pešuṭim to a third and a quarter without a remainder | ||
:<math>\scriptstyle\frac{1}{3}X+\frac{1}{4}X=5</math> | :<math>\scriptstyle\frac{1}{3}X+\frac{1}{4}X=5</math> | ||
| − | |style="text-align:right;"|נד | + | |style="text-align:right;"|נד <big>אם</big> תרצה לחלק ה"פ לשליש ולרביע ולא ישאר ממנו כלל{{#annotend:q2Sp}} |
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''False Position - common denominator:'''</span> First, say: a third and a quarter are found in 12. | |
|style="text-align:right;"|אמור תחלה שליש ורביע נמצא בי"ב | |style="text-align:right;"|אמור תחלה שליש ורביע נמצא בי"ב | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\frac{1}{3}\sdot12=4}}</math> | + | :The third is 4. |
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{3}\sdot12=4}}</math> | ||
|style="text-align:right;"|השליש הוא ד‫' | |style="text-align:right;"|השליש הוא ד‫' | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\frac{1}{4}\sdot12=3}}</math> | + | :The quarter is 3. |
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{4}\sdot12=3}}</math> | ||
|style="text-align:right;"|הרביע הוא ג‫' | |style="text-align:right;"|הרביע הוא ג‫' | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Denominator:'''</span> Sum them together; they are 7 and this is the denominator. | |
| + | :<math>\scriptstyle{\color{blue}{4+3=7}}</math> | ||
|style="text-align:right;"|חברם יחד הם ז' והוא המחלק | |style="text-align:right;"|חברם יחד הם ז' והוא המחלק | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> If you want to know how much is the portion of the one who has a third, which is 4, say: 4 times 5, since they are 5 pešuṭim; the result is 20. Divide them by 7; the result is 2 and 6 parts of 7. | |
| − | + | :<math>\scriptstyle{\color{blue}{\frac{1}{3}X=\frac{4\sdot5}{7}=\frac{20}{7}=2+\frac{6}{7}}}</math> | |
|style="text-align:right;"|ואם תרצה לידע כמה יבא למי שיש לו השליש שהוא ד‫'<br> | |style="text-align:right;"|ואם תרצה לידע כמה יבא למי שיש לו השליש שהוא ד‫'<br> | ||
אמור ד' פעמי' ה' בעבור שהם הפ' יבא כ‫'<br> | אמור ד' פעמי' ה' בעבור שהם הפ' יבא כ‫'<br> | ||
| Line 1,800: | Line 2,137: | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> For the one who has a quarter, which is 3, say: 3 times 5 is 15. Divide them by 7; the result is 2 and one part of 7. | |
| − | + | :<math>\scriptstyle{\color{blue}{\frac{1}{4}X=\frac{3\sdot5}{7}=\frac{15}{7}=2+\frac{1}{7}}}</math> | |
|style="text-align:right;"|ומי שהוא שיש לו הרביע שהוא ג‫'<br> | |style="text-align:right;"|ומי שהוא שיש לו הרביע שהוא ג‫'<br> | ||
אמור ג' פעמי' ה' ט"ו<br> | אמור ג' פעמי' ה' ט"ו<br> | ||
| Line 1,807: | Line 2,144: | ||
|- | |- | ||
| | | | ||
| − | :Check: <math>\scriptstyle{\color{blue}{\left(2+\frac{6}{7}\right)+\left(2+\frac{1}{7}\right)=5}}</math> | + | :<span style=color:green>'''Check:'''</span> Sum them together; the result is 5, as the amount of money, since they are divided into a third and a quarter and there is nothing left of them. |
| − | |style="text-align:right;"|חברם יחד יעלו ה' | + | :<math>\scriptstyle{\color{blue}{\left(2+\frac{6}{7}\right)+\left(2+\frac{1}{7}\right)=5}}</math> |
| + | |style="text-align:right;"|חברם יחד יעלו ה' כמספר המעות הרי ‫<ref>93r</ref>שנחלקו לשליש ולרביע ולא נשאר מהם כלל וכה"ל | ||
|- | |- | ||
| − | | | + | | |
| + | |||
| + | === <span style=color:green>Divide a Quantity – Money</span> === | ||
| + | |||
| | | | ||
|- | |- | ||
| Line 1,816: | Line 2,157: | ||
:{{#annot:money|645|Ikua}}55) If you want to divide 12 pešuṭim to a half, a third, and a quarter without a remainder | :{{#annot:money|645|Ikua}}55) If you want to divide 12 pešuṭim to a half, a third, and a quarter without a remainder | ||
:<math>\scriptstyle\frac{1}{2}X+\frac{1}{3}X+\frac{1}{4}X=12</math> | :<math>\scriptstyle\frac{1}{2}X+\frac{1}{3}X+\frac{1}{4}X=12</math> | ||
| − | |style="text-align:right;"|נה | + | |style="text-align:right;"|נה <big>אם</big> תרצה לחלק י"ב פשוט לחצי ולשליש ולרביע{{#annotend:Ikua}} |
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''False Position - common denominator:'''</span> First say where they are found - in 12. | |
|style="text-align:right;"|אמור תחלה אנה ימצאו בי"ב | |style="text-align:right;"|אמור תחלה אנה ימצאו בי"ב | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\frac{1}{2}\sdot12=6}}</math> | + | :The half is 6. |
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{2}\sdot12=6}}</math> | ||
|style="text-align:right;"|החצי הוא ו‫' | |style="text-align:right;"|החצי הוא ו‫' | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\frac{1}{3}\sdot12=4}}</math> | + | :The third is 4. |
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{3}\sdot12=4}}</math> | ||
|style="text-align:right;"|והשליש ד‫' | |style="text-align:right;"|והשליש ד‫' | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\frac{1}{4}\sdot12=3}}</math> | + | :The quarter is 3. |
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{4}\sdot12=3}}</math> | ||
|style="text-align:right;"|והרביע ג‫' | |style="text-align:right;"|והרביע ג‫' | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Denominator:'''</span> Sum them; they are 13 and this is the denominator. | |
| − | + | :<math>\scriptstyle{\color{blue}{6+4+3=13}}</math> | |
|style="text-align:right;"|חברם הם י"ג והוא המחלק | |style="text-align:right;"|חברם הם י"ג והוא המחלק | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> For the one who has a half, say: the half of 12 is 6. Say: 6 times 12, for the amount of money, which is 12; the result is 72. Divide it by 13; the result is 5 and 7 parts of 13. | |
| − | + | :<math>\scriptstyle{\color{blue}{\frac{1}{2}X=\frac{6\sdot12}{13}=\frac{72}{13}=5+\frac{7}{13}}}</math> | |
|style="text-align:right;"|ומי שיש לו החצי אמור החצי מי"ב הוא ו‫'<br> | |style="text-align:right;"|ומי שיש לו החצי אמור החצי מי"ב הוא ו‫'<br> | ||
ואמור ו' פעמי' י"ב בעבור המעות שהם י"ב יבואו ע"ב<br> | ואמור ו' פעמי' י"ב בעבור המעות שהם י"ב יבואו ע"ב<br> | ||
| Line 1,847: | Line 2,191: | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> For the one who has a third, which is 4, say: 4 times 12 is 48. Divide it by 13; the result is 3 and 9 parts of 13. | |
| − | + | :<math>\scriptstyle{\color{blue}{\frac{1}{3}X=\frac{4\sdot12}{13}=\frac{48}{13}=3+\frac{9}{13}}}</math> | |
|style="text-align:right;"|ומי שיש לו השליש שהוא ד‫'<br> | |style="text-align:right;"|ומי שיש לו השליש שהוא ד‫'<br> | ||
אמור ד' י"ב הם מ"ח<br> | אמור ד' י"ב הם מ"ח<br> | ||
| Line 1,854: | Line 2,198: | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> For the one who has a quarter, which is 3, say: 3 times 12 is 36. Divide it by 13; the result is 2 and 10 parts of 13. | |
| − | + | :<math>\scriptstyle{\color{blue}{\frac{1}{4}X=\frac{3\sdot12}{13}=\frac{36}{13}=2+\frac{10}{13}}}</math> | |
|style="text-align:right;"|ומי שיש לו הרביע שהוא ג‫'<br> | |style="text-align:right;"|ומי שיש לו הרביע שהוא ג‫'<br> | ||
אמור ג' פעמי' י"ב הם ל"ו<br> | אמור ג' פעמי' י"ב הם ל"ו<br> | ||
| Line 1,861: | Line 2,205: | ||
|- | |- | ||
| | | | ||
| − | :Check: <math>\scriptstyle{\color{blue}{\left(5+\frac{7}{13}\right)+\left(3+\frac{9}{13}\right)+\left(2+\frac{10}{13}\right)=12}}</math> | + | :<span style=color:green>'''Check:'''</span> If you sum all of them, the result is 12 pešuṭim as the amount of money. |
| + | :<math>\scriptstyle{\color{blue}{\left(5+\frac{7}{13}\right)+\left(3+\frac{9}{13}\right)+\left(2+\frac{10}{13}\right)=12}}</math> | ||
|style="text-align:right;"|ואם תאסוף כלם יבאו יב"פ כמספ' המעו‫' | |style="text-align:right;"|ואם תאסוף כלם יבאו יב"פ כמספ' המעו‫' | ||
|- | |- | ||
| − | | | + | | |
| + | |||
| + | === <span style=color:green>Ordering Problem - Six Coins</span> === | ||
| + | |||
| | | | ||
|- | |- | ||
| Line 1,873: | Line 2,221: | ||
:How much should [each of] the six coins [be worth] so that he will not need to exchange them? | :How much should [each of] the six coins [be worth] so that he will not need to exchange them? | ||
:<math>\scriptstyle{\color{blue}{1+2+3+4+8+12=30}}</math> | :<math>\scriptstyle{\color{blue}{1+2+3+4+8+12=30}}</math> | ||
| − | |style="text-align:right;"|נו | + | |style="text-align:right;"|נו <big>הרי</big> שאדם יש לו שכיר אחד לשלשי' יום בל' פשו‫'<br> |
ורוצה לקבל פרעונו בכל יום ואין לשוכר רק ו' מטבעות כסף ששוים ל"פ סך כולם<br> | ורוצה לקבל פרעונו בכל יום ואין לשוכר רק ו' מטבעות כסף ששוים ל"פ סך כולם<br> | ||
והוא פורע אותו בכל יום עם אלו הו' מטבעות<br> | והוא פורע אותו בכל יום עם אלו הו' מטבעות<br> | ||
| Line 1,879: | Line 2,227: | ||
|- | |- | ||
| | | | ||
| − | ::1; 2; 3; 4; 8; 12 | + | :The signs: 1; 2; 3; 4; 8; 12. |
|style="text-align:right;"|סימ' א' ב' ג' ד' ח' י"ב | |style="text-align:right;"|סימ' א' ב' ג' ד' ח' י"ב | ||
|- | |- | ||
| − | | | + | | |
| + | |||
| + | === <span style=color:green>Find a Number Problem - Ratio of Integers and Fractions</span> === | ||
| + | |||
| | | | ||
|- | |- | ||
| Line 1,888: | Line 2,239: | ||
:57) {{#annot:3⅓÷4¼=5⅕÷X|567|Epgk}}If three and one third equal four and a quarter, how much are five and one fifth equal? | :57) {{#annot:3⅓÷4¼=5⅕÷X|567|Epgk}}If three and one third equal four and a quarter, how much are five and one fifth equal? | ||
:<math>\scriptstyle\left(3+\frac{1}{3}\right):\left(4+\frac{1}{4}\right)=\left(5+\frac{1}{5}\right):X</math> | :<math>\scriptstyle\left(3+\frac{1}{3}\right):\left(4+\frac{1}{4}\right)=\left(5+\frac{1}{5}\right):X</math> | ||
| − | |style="text-align:right;"|נז | + | |style="text-align:right;"|נז <big>אם</big> ג' ושליש שוים ד' ורביע ה' וחומש כמה שוים{{#annotend:Epgk}} |
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{X=\frac{\left(5+\frac{1}{5}\right)\sdot\left(4+\frac{1}{4}\right)}{3+\frac{1}{3}}}}</math> | + | :Say: 5 and a fifth times 4 and a quarter; then divide the result by 3 and a third. |
| + | :<math>\scriptstyle{\color{blue}{X=\frac{\left(5+\frac{1}{5}\right)\sdot\left(4+\frac{1}{4}\right)}{3+\frac{1}{3}}}}</math> | ||
|style="text-align:right;"|אמור ה' וחומש פעמי' ד' ורביע ומה שיבא חלקהו בג' ושליש | |style="text-align:right;"|אמור ה' וחומש פעמי' ד' ורביע ומה שיבא חלקהו בג' ושליש | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{5+\frac{1}{5}=\frac{\left(5\sdot5\right)+1}{5}=\frac{25+1}{5}=\frac{26}{5}}}</math> | + | :This is the order of the procedure: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וזה סדר עשייתו |
| + | |- | ||
| + | | | ||
| + | :Take 5 and a fifth and say: 5 times [5] is 25; with 2 it is 26. So, you have converted 5 and a fifth into 26-fifths, as you see. | ||
| + | :<math>\scriptstyle{\color{blue}{5+\frac{1}{5}=\frac{\left(5\sdot5\right)+1}{5}=\frac{25+1}{5}=\frac{26}{5}}}</math> | ||
| + | |style="text-align:right;"|תפוש ה' וחומש ואמור ה' פעמי' כ"ה וא' הרי כ"ו<br> | ||
הרי שעשית מה' וחומש כ"ו חמשיות ותזמן אותם כאשר תראה | הרי שעשית מה' וחומש כ"ו חמשיות ותזמן אותם כאשר תראה | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{4+\frac{1}{4}=\frac{\left(4\sdot4\right)+1}{4}=\frac{16+1}{4}=\frac{17}{4}}}</math> | + | :Then, take 4 and a quarter and say: 4 times 4 is 16; with 1 it is 17. So, you have converted 4 and a quarter into 17-quarters. |
| + | :<math>\scriptstyle{\color{blue}{4+\frac{1}{4}=\frac{\left(4\sdot4\right)+1}{4}=\frac{16+1}{4}=\frac{17}{4}}}</math> | ||
|style="text-align:right;"|אחרי כן תפוש ד' ורביע ואמור ד' פעמי' ד' י"ו וא' הרי י"ז<br> | |style="text-align:right;"|אחרי כן תפוש ד' ורביע ואמור ד' פעמי' ד' י"ו וא' הרי י"ז<br> | ||
הרי שעשית מד' ורביע י"ז רבעיות | הרי שעשית מד' ורביע י"ז רבעיות | ||
|- | |- | ||
| | | | ||
| − | :: | + | :Since we must say: 5 and a fifth times 4 and a quarter, multiply 26 by 17; the result is 442. Then, we divide them into a quarter and a fifth and say: 4 times 5 is 20. We divide 442 by it; the result is 22 and 2 parts of 20, i.e. one tenth. |
|style="text-align:right;"|ובעבור שאנו צריכים לומ' ה' וחומש פעמי' ד' ורביע תרבה כ"ו עם י"ז יעלו תמ"ב<br> | |style="text-align:right;"|ובעבור שאנו צריכים לומ' ה' וחומש פעמי' ד' ורביע תרבה כ"ו עם י"ז יעלו תמ"ב<br> | ||
ונחלק אותם אחרי כן לרביע וחומש ונאמ' ד' פעמי' ה' כ‫'<br> | ונחלק אותם אחרי כן לרביע וחומש ונאמ' ד' פעמי' ה' כ‫'<br> | ||
| − | נחלק אותם תמ"ב יעלו כ"ב וב' חלקי' מכ' דהיינו א' עשירי‫' | + | נחלק אותם [תמ]"ב יעלו כ"ב וב' חלקי' מכ' דהיינו א' עשירי‫' |
| + | |- | ||
| + | | colspan="2"| | ||
| + | :<math>\scriptstyle{\color{blue}{\left(5+\frac{1}{5}\right)\sdot\left(4+\frac{1}{4}\right)=\frac{26}{5}\sdot\frac{17}{4}=\frac{26\sdot17}{5\sdot4}=\frac{442}{20}=22+\frac{2}{20}=22+\frac{1}{10}}}</math> | ||
|- | |- | ||
| | | | ||
| − | : | + | :Since we must divide by 3 and a third, convert 22 and a tenth into thirds and say: 3 times 22 is 66; 3 times a tenth is 3-tenths. So, they are 66 and 3-tenths. |
| − | + | :<math>\scriptstyle{\color{blue}{\left(5+\frac{1}{5}\right)\sdot\left(4+\frac{1}{4}\right)=22+\frac{1}{10}=\frac{3\sdot\left(22+\frac{1}{10}\right)}{3}=\frac{66+\frac{3}{10}}{3}}}</math> | |
|style="text-align:right;"|ובעבור שאנו צריכים לחלק בג' ושליש עשה מכ"ב וא' עשירי' כולם שלישייות ואמור ג' פעמי' כ"ב ס"ו וג' פעמי' א' עשירי' הם ג' עשריות הרי ס"ו וג' עשירייות | |style="text-align:right;"|ובעבור שאנו צריכים לחלק בג' ושליש עשה מכ"ב וא' עשירי' כולם שלישייות ואמור ג' פעמי' כ"ב ס"ו וג' פעמי' א' עשירי' הם ג' עשריות הרי ס"ו וג' עשירייות | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{3+\frac{1}{3}=\frac{\left(3\sdot3\right)+1}{3}=\frac{9+1}{3}=\frac{10}{3}}}</math> | + | :Then, divide them by 3 and a third and this is the division procedure: convert 3 and a third into thirds and say: 3 times 3 is 9; with 1 it is 10. |
| + | :<math>\scriptstyle{\color{blue}{3+\frac{1}{3}=\frac{\left(3\sdot3\right)+1}{3}=\frac{9+1}{3}=\frac{10}{3}}}</math> | ||
|style="text-align:right;"|חלק אותם אחרי כן בג' ושליש וזהו דרך חלוקו שתעשה מג' ושליש כולם שלישייות ואמור ג' פעמי' ג' ט' וא' הרי י‫' | |style="text-align:right;"|חלק אותם אחרי כן בג' ושליש וזהו דרך חלוקו שתעשה מג' ושליש כולם שלישייות ואמור ג' פעמי' ג' ט' וא' הרי י‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | :We divide 66 into 10 parts; the result is 6 and 6-tenths. |
| − | |style="text-align:right;"|א"כ נחלק ס"ו בי' חלקי' יבואו ו' וו' עשירייות | + | |style="text-align:right;"|א"כ נחלק ס"ו בי' חלקי' יבואו ו' וו' עשירייות |
| − | נחלק אחרי כן ג' עשיריות בי' חלקי' יבואו' ג' חלקי' מק‫' | + | |- |
| − | הרי שעולה החשבון ו' שלמים וו' עשירייות וג' חלקי' מק‫' | + | | |
| − | נקבץ אותם יחד ונעשה מו' עשיריות חלקי' מק' ויהיו ס' וג' חלקי' שהיו לנו הרי ס"ג<br> | + | :We divide 3-tenths into 10 parts; the result is 3 parts of 100. |
| + | |style="text-align:right;"|נחלק אחרי כן ג' עשיריות בי' חלקי' יבואו' ג' חלקי' מק‫' | ||
| + | |- | ||
| + | | | ||
| + | :The result is 6 integers, 6-tenths and 3 parts of 100. | ||
| + | |style="text-align:right;"|הרי שעולה החשבון ו' שלמים וו' עשירייות וג' חלקי' מק‫' | ||
| + | |- | ||
| + | | | ||
| + | :We sum them together and convert the 6-tenths into parts of 100; they are 60; with the 3 parts we had they are 63. We find that the result is 6 integers and 63 parts of one hundred. | ||
| + | |style="text-align:right;"|נקבץ אותם יחד ונעשה מו' עשיריות חלקי' מק' ויהיו ס' וג' חלקי' שהיו לנו הרי ס"ג<br> | ||
נמצא שיבא ו' שלמים וס"ג חלקי' ממאה | נמצא שיבא ו' שלמים וס"ג חלקי' ממאה | ||
|- | |- | ||
| − | | | + | | colspan="2"| |
| + | :<math>\scriptstyle{\color{blue}{X=\frac{\left(5+\frac{1}{5}\right)\sdot\left(4+\frac{1}{4}\right)}{3+\frac{1}{3}}=\frac{\frac{66+\frac{3}{10}}{3}}{\frac{10}{3}}=\frac{66+\frac{3}{10}}{10}=\frac{66}{10}+\frac{\frac{3}{10}}{10}=6+\frac{6}{10}+\frac{3}{100}=6+\frac{60}{100}+\frac{3}{100}=6+\frac{63}{100}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | === <span style=color:green>Multiple Quantities - Boys Selling Eggs</span> === | ||
| + | |||
| | | | ||
|- | |- | ||
| Line 1,934: | Line 2,311: | ||
:He said to them: go, sell the eggs equally, and bring me equal amounts of money | :He said to them: go, sell the eggs equally, and bring me equal amounts of money | ||
:<math>\scriptstyle10X=30Y=50Z</math> | :<math>\scriptstyle10X=30Y=50Z</math> | ||
| − | |style="text-align:right;"|נח | + | |style="text-align:right;"|נח <big>אדם</big> נתן לבניו ביצות למכור לאחד נתן נ' בצים ולשני ל' ולשלישי י‫'<br> |
ואמ' להם לכו ומכרו הבצים בשוה וכולכם תביאו לי מעות בשוה{{#annotend:f4Im}} | ואמ' להם לכו ומכרו הבצים בשוה וכולכם תביאו לי מעות בשוה{{#annotend:f4Im}} | ||
|- | |- | ||
| | | | ||
| − | : | + | :[First], they sold 7 eggs for one pašuṭ: |
|style="text-align:right;"|הלכו ונתנו ז' בצים בפשוט | |style="text-align:right;"|הלכו ונתנו ז' בצים בפשוט | ||
|- | |- | ||
| | | | ||
| − | : | + | :The one who had 50 eggs sold 49 eggs for 7 pešuṭim and had one egg left. |
| − | + | :<math>\scriptstyle{\color{blue}{50-\left(7\sdot7\right)=50-49=1}}</math> | |
|style="text-align:right;"|אותו שהיו לו נ' בצים מכר מ"ט בצי' בז"פ ונשארה לו ביצה אחת | |style="text-align:right;"|אותו שהיו לו נ' בצים מכר מ"ט בצי' בז"פ ונשארה לו ביצה אחת | ||
|- | |- | ||
| | | | ||
| − | : | + | :The one who had 30 eggs sold 28 eggs for 4 pešuṭim and had 2 eggs left. |
| − | + | :<math>\scriptstyle{\color{blue}{30-\left(7\sdot4\right)=30-28=2}}</math> | |
|style="text-align:right;"|ואותו שהיו לו ל' בצים מכר כ"ח בצים בד' פשו' ונשארו לו ב' בצים | |style="text-align:right;"|ואותו שהיו לו ל' בצים מכר כ"ח בצים בד' פשו' ונשארו לו ב' בצים | ||
|- | |- | ||
| | | | ||
| − | : | + | :The one who had 10 eggs sold 7 eggs for one pašuṭ and had 3 eggs left. |
| − | + | :<math>\scriptstyle{\color{blue}{10-\left(7\sdot1\right)=10-7=3}}</math> | |
|style="text-align:right;"|ואותו שהיו לו י' בצים מכר ז' בצים בפשו' ונשארו לו ג' בצים | |style="text-align:right;"|ואותו שהיו לו י' בצים מכר ז' בצים בפשו' ונשארו לו ג' בצים | ||
|- | |- | ||
| | | | ||
| − | : | + | :Then, they sold the eggs they had left at 3 pešuṭim for an egg: |
|style="text-align:right;"|הילכו פעם שנייה ומכרו הבצים שנשארו להם בג"פ הביצה | |style="text-align:right;"|הילכו פעם שנייה ומכרו הבצים שנשארו להם בג"פ הביצה | ||
|- | |- | ||
| | | | ||
| − | : | + | :The first who had one egg left received 3 pešuṭim; with the 7 he received from the first sale they are 10 pešuṭim. |
| − | : | + | :<math>\scriptstyle{\color{blue}{\left(1\sdot3\right)+7=3+7=10}}</math> |
|style="text-align:right;"|הראשון שנשארה לו ביצה אחת קבל ג' פשוט וז' שקבל במכירה ראשונה הרי י' פשו‫' | |style="text-align:right;"|הראשון שנשארה לו ביצה אחת קבל ג' פשוט וז' שקבל במכירה ראשונה הרי י' פשו‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | :The second who had 2 eggs left received 6 pešuṭim; with the 4 pešuṭim he received from the first sale they are 10 pešuṭim. |
| − | : | + | :<math>\scriptstyle{\color{blue}{\left(2\sdot3\right)+4=6+4=10}}</math> |
|style="text-align:right;"|והשני שנשארו לו ב' בצים קבל ו"פ וד"פ שקבל במכירה ראשונה הרי י"פ | |style="text-align:right;"|והשני שנשארו לו ב' בצים קבל ו"פ וד"פ שקבל במכירה ראשונה הרי י"פ | ||
|- | |- | ||
| | | | ||
| − | : | + | :The third who had 3 eggs left received 9 pešuṭim; with the 1 pešuṭ he received from the first sale he got 10 pešuṭim. |
| − | : | + | :<math>\scriptstyle{\color{blue}{\left(3\sdot3\right)+1=9+1=10}}</math> |
| − | |style="text-align:right;"|והשלישי שנשארו לו ג' בצים קבל ט"פ וא"פ שקבל במכירה ראשונה הרי שקבל י"פ | + | |style="text-align:right;"|‫<ref>93v</ref>והשלישי שנשארו לו ג' בצים קבל ט"פ וא"פ שקבל במכירה ראשונה הרי שקבל י"פ |
|- | |- | ||
| | | | ||
| − | : | + | :We find that they sold the eggs for the same price and received the same amount of money. |
|style="text-align:right;"|נמצא שכולם מכרו הבצים בשוה וכולם קיבלו מעות בשוה | |style="text-align:right;"|נמצא שכולם מכרו הבצים בשוה וכולם קיבלו מעות בשוה | ||
| − | |||
| − | |||
|- | |- | ||
| − | + | | | |
| + | |||
| + | === <span style=color:green>Find a Quantity Problem - Whole from Parts – Fish</span> === | ||
| + | |||
| | | | ||
|- | |- | ||
| Line 1,991: | Line 2,369: | ||
:How much does the whole fish weigh? | :How much does the whole fish weigh? | ||
:<math>\scriptstyle\frac{1}{3}X+\frac{1}{4}X+\frac{1}{5}X+\frac{1}{6}X+10=X</math> | :<math>\scriptstyle\frac{1}{3}X+\frac{1}{4}X+\frac{1}{5}X+\frac{1}{6}X+10=X</math> | ||
| − | |style=" | + | |style="text-align:right;"|נט <big>הרי</big> שיש לפניך דג ונחתך ראשו וזנבו<br> |
והגוף שוקל י' ליט‫'<br> | והגוף שוקל י' ליט‫'<br> | ||
והראש היה שוקל השליש והרביע מכל הדג<br> | והראש היה שוקל השליש והרביע מכל הדג<br> | ||
| Line 1,998: | Line 2,376: | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''False Position:'''</span> First say: a third, a quarter, a fifth, and a sixth are found in 60. | |
|style="text-align:right;"|אמור תחלה שליש ורביע וחומש ושתות נמצא בס‫' | |style="text-align:right;"|אמור תחלה שליש ורביע וחומש ושתות נמצא בס‫' | ||
|- | |- | ||
| | | | ||
| − | + | :They are 20, 15, 12, and 10, which are 57 parts of 60. | |
|style="text-align:right;"|שהם כ' וט"ו וי"ב וי' שהם נ"ז חלקי' מס‫' | |style="text-align:right;"|שהם כ' וט"ו וי"ב וי' שהם נ"ז חלקי' מס‫' | ||
|- | |- | ||
| | | | ||
| − | + | :We find that the head and tail that weigh together a third, a quarter, a fifth, and a sixth are 57 parts of 60, which is the whole. | |
| − | |||
|style="text-align:right;"|נמצא שהראש והזנב ששקלו סך שניהם שליש ורביע וחומש ושתות הם נ"ז חלקי' מס' שהוא השלם | |style="text-align:right;"|נמצא שהראש והזנב ששקלו סך שניהם שליש ורביע וחומש ושתות הם נ"ז חלקי' מס' שהוא השלם | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | :<math>\scriptstyle{\color{blue}{\frac{\left(\frac{1}{3}\sdot60\right)+\left(\frac{1}{4}\sdot60\right)+\left(\frac{1}{5}\sdot60\right)+\left(\frac{1}{6}\sdot60\right)}{60}=\frac{20+15+12+10}{60}=\frac{57}{60}}}</math> | ||
|- | |- | ||
| | | | ||
| − | + | :The 3 parts that remain from 60 are the body that weighs 10 liṭra. | |
| − | + | :<math>\scriptstyle{\color{blue}{\frac{60}{60}-\frac{57}{60}=\frac{3}{60}}}</math> | |
|style="text-align:right;"|ואותם הג' חלקי' שנשארו עד ס' הוא הגוף ששוקל י' ליט‫' | |style="text-align:right;"|ואותם הג' חלקי' שנשארו עד ס' הוא הגוף ששוקל י' ליט‫' | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> So say: if 3 parts of 60, i.e. the body, weigh 10 liṭra, how much do the remaining 57 parts of 60, i.e. the third, the quarter, the fifth, and the sixth, which are the head and the tail weigh? | |
| − | + | :<math>\scriptstyle{\color{blue}{\frac{3}{60}:10=\frac{57}{60}:a}}</math> | |
|style="text-align:right;"|אמור א"כ אם ג' חלקי' מס' שוקלים י' ליט' דהיינו הגוף הנשאר הנ"ז חלקי' מס' דהיינו השליש והרביע והחומש ושתות שהם הראש והזנב כמה שוקלי‫' | |style="text-align:right;"|אמור א"כ אם ג' חלקי' מס' שוקלים י' ליט' דהיינו הגוף הנשאר הנ"ז חלקי' מס' דהיינו השליש והרביע והחומש ושתות שהם הראש והזנב כמה שוקלי‫' | ||
|- | |- | ||
| | | | ||
| − | + | :Say: 57 times 10 is 570. | |
|style="text-align:right;"|אמור נ"ז פעמי' י' הם תק"ע | |style="text-align:right;"|אמור נ"ז פעמי' י' הם תק"ע | ||
|- | |- | ||
| | | | ||
| − | + | :Divide it by 3 parts; the result is 190 liṭra. | |
| − | + | :<math>\scriptstyle{\color{blue}{a=\frac{57\sdot10}{3}=\frac{570}{3}=190}}</math> | |
|style="text-align:right;"|חלקם בג' חלקי' יבוא ק"צ ליט‫' | |style="text-align:right;"|חלקם בג' חלקי' יבוא ק"צ ליט‫' | ||
|- | |- | ||
| | | | ||
| − | + | :We find that the head and the tail weigh 190 liṭra. | |
|style="text-align:right;"|נמצא שהראש והזנב שוקלים ק"צ ליט‫' | |style="text-align:right;"|נמצא שהראש והזנב שוקלים ק"צ ליט‫' | ||
|- | |- | ||
| | | | ||
| − | + | :The body weighs 10 liṭra, so the total is 200 liṭra. | |
| − | + | :<math>\scriptstyle{\color{blue}{x=190+10=200}}</math> | |
|style="text-align:right;"|והגוף שוקל י' ליט' שהוא סך הכל ר' ליט‫' | |style="text-align:right;"|והגוף שוקל י' ליט' שהוא סך הכל ר' ליט‫' | ||
|- | |- | ||
| | | | ||
| − | + | :If you wish to know how much does the head weigh alone: | |
|style="text-align:right;"|ואם תרצה לידע כמה שוקל הראש לבדו | |style="text-align:right;"|ואם תרצה לידע כמה שוקל הראש לבדו | ||
|- | |- | ||
| | | | ||
| − | + | :You already know that the head weighs a third and a quarter; and the third and the quarter of 60 are 35 parts of 60. | |
| − | + | :<math>\scriptstyle{\color{blue}{\frac{\left(\frac{1}{3}\sdot60\right)+\left(\frac{1}{4}\sdot60\right)}{60}=\frac{20+15}{60}=\frac{35}{60}}}</math> | |
|style="text-align:right;"|כבר ידעת כי הראש שוקל השליש והרביע והשליש והרביע מס' הם ל"ה חלקי' מס‫' | |style="text-align:right;"|כבר ידעת כי הראש שוקל השליש והרביע והשליש והרביע מס' הם ל"ה חלקי' מס‫' | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> Say: if 3 parts of 60, i.e. the body, weigh 10 liṭra, how much do these 35 parts, i.e. the head that weighs a third and a quarter, weigh? | |
| − | + | :<math>\scriptstyle{\color{blue}{\frac{3}{60}:10=\frac{35}{60}:b}}</math> | |
|style="text-align:right;"|ואמור אם ג' חלקי' מס' דהיינו הגוף שוקל י' ליט' אלו הל"ה חלקי' דהיינו הראש ששוקל שליש ורביע כמה שוקל | |style="text-align:right;"|ואמור אם ג' חלקי' מס' דהיינו הגוף שוקל י' ליט' אלו הל"ה חלקי' דהיינו הראש ששוקל שליש ורביע כמה שוקל | ||
|- | |- | ||
| | | | ||
| − | + | :Say 35 times 10 [is] 350. | |
|style="text-align:right;"|אמור ל"ה פעמי' י' ש"נ | |style="text-align:right;"|אמור ל"ה פעמי' י' ש"נ | ||
|- | |- | ||
| | | | ||
| − | + | :Divide it by 3; the result is 116 and 2-thirds and this is the weight of the head. | |
| − | + | :<math>\scriptstyle{\color{blue}{b=\frac{35\sdot10}{3}=\frac{350}{3}=116+\frac{2}{3}}}</math> | |
|style="text-align:right;"|חלקם בג' יבא קי"ו וב' שלשיות והוא משקל הראש | |style="text-align:right;"|חלקם בג' יבא קי"ו וב' שלשיות והוא משקל הראש | ||
|- | |- | ||
| | | | ||
| − | + | :Do the same with the tail and you will get the matter. | |
| − | |style="text-align:right;"|ובזה הדרך תעשה גם הזנב ויצא לך הענין | + | |style="text-align:right;"|ובזה הדרך תעשה גם הזנב ויצא לך הענין וכה"ל |
|- | |- | ||
| | | | ||
| − | + | ||
| − | + | === <span style=color:green>Simultaneous Division - Money in a Purse</span> === | |
| − | + | ||
| − | |||
| − | |||
| | | | ||
|- | |- | ||
| Line 2,079: | Line 2,457: | ||
:How much money did each one of them have? | :How much money did each one of them have? | ||
:<math>\scriptstyle\frac{1}{3}X+\frac{1}{4}X=\frac{1}{6}Y+\frac{1}{7}Y</math> | :<math>\scriptstyle\frac{1}{3}X+\frac{1}{4}X=\frac{1}{6}Y+\frac{1}{7}Y</math> | ||
| − | |style="text-align:right;"|ס | + | |style="text-align:right;"|ס <big>ב</big> אנשים היו להם מעות בא' כיס<br> |
אמ' זה לזה שמעני אחי כך הם השליש והרביע ממעותי כמו השתות והשבע ממעותיך<br> | אמ' זה לזה שמעני אחי כך הם השליש והרביע ממעותי כמו השתות והשבע ממעותיך<br> | ||
והם בדקו ומצאו כן כאשר אמרו<br> | והם בדקו ומצאו כן כאשר אמרו<br> | ||
| Line 2,085: | Line 2,463: | ||
|- | |- | ||
| | | | ||
| − | : | + | :Find the number that has a third and a quarter; they are found in 12 and this is the amount of money of he had. |
|style="text-align:right;"|תמצא חשבון שימצא בו שליש ורביע וימצא בי"ב וכך מעות היו לו | |style="text-align:right;"|תמצא חשבון שימצא בו שליש ורביע וימצא בי"ב וכך מעות היו לו | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\left(\frac{1}{3}\sdot12\right)+\left(\frac{1}{4}\sdot12\right)=7}}</math> | + | :Take a third and a quarter of it; they are 7 pešuṭim. |
| + | :<math>\scriptstyle{\color{blue}{\left(\frac{1}{3}\sdot12\right)+\left(\frac{1}{4}\sdot12\right)=7}}</math> | ||
|style="text-align:right;"|קח מהם שליש ורביע והם ז"פ | |style="text-align:right;"|קח מהם שליש ורביע והם ז"פ | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\frac{\left(\frac{1}{6}\sdot42\right)+\left(\frac{1}{7}\sdot42\right)}{42}=\frac{13}{42}}}</math> | + | :For the other who had a sixth and a seventh that are 13 parts of 42: |
| + | :<math>\scriptstyle{\color{blue}{\frac{\left(\frac{1}{6}\sdot42\right)+\left(\frac{1}{7}\sdot42\right)}{42}=\frac{13}{42}}}</math> | ||
|style="text-align:right;"|ועבור האיש האחר שהיה לו שתות ושביע שהם י"ג חלקי' ממ"ב | |style="text-align:right;"|ועבור האיש האחר שהיה לו שתות ושביע שהם י"ג חלקי' ממ"ב | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> say: if 13 are 7, how much is 42? | |
| + | :<math>\scriptstyle{\color{blue}{13:7=42:y}}</math> | ||
|style="text-align:right;"|ואמור אם י"ג היו ז' כמה היו מ"ב | |style="text-align:right;"|ואמור אם י"ג היו ז' כמה היו מ"ב | ||
|- | |- | ||
| | | | ||
| − | ::the money of | + | :Say: 7 times 42 is 294. Divide it by 13; it is 22 and 8 parts of 13 and this is the amount of money of he had. |
| + | :<math>\scriptstyle{\color{blue}{y=\frac{7\sdot42}{13}=\frac{294}{13}=22+\frac{8}{13}}}</math> | ||
|style="text-align:right;"|אמור ז' פעמי' מ"ב רצ"ד<br> | |style="text-align:right;"|אמור ז' פעמי' מ"ב רצ"ד<br> | ||
חלקם בי"ג יהיו כ"ב וח' חלקי' מי"ג וכך מעות היו לו | חלקם בי"ג יהיו כ"ב וח' חלקי' מי"ג וכך מעות היו לו | ||
|- | |- | ||
| | | | ||
| − | :Check: <math>\scriptstyle{\color{blue}{\left[\frac{1}{6}\sdot\left(22+\frac{8}{13}\right)\right]+\left[\frac{1}{7}\sdot\left(22+\frac{8}{13}\right)\right]=7}}</math> | + | :<span style=color:green>'''Check:'''</span> Take a sixth and a seventh of it; they are 7 pešuṭim. |
| + | :<math>\scriptstyle{\color{blue}{\left[\frac{1}{6}\sdot\left(22+\frac{8}{13}\right)\right]+\left[\frac{1}{7}\sdot\left(22+\frac{8}{13}\right)\right]=7}}</math> | ||
|style="text-align:right;"|תוציא השתות והשביע מהם ויהיו ז"פ | |style="text-align:right;"|תוציא השתות והשביע מהם ויהיו ז"פ | ||
|- | |- | ||
| − | | | + | | |
| + | |||
| + | === <span style=color:green>Partnership - for the same time</span> === | ||
| + | |||
| | | | ||
|- | |- | ||
| Line 2,119: | Line 2,505: | ||
:How much gold should each of them contribute so that the total will be 40 ounces and the share of each of them will be equal to the shares of his friends? | :How much gold should each of them contribute so that the total will be 40 ounces and the share of each of them will be equal to the shares of his friends? | ||
:<math>\scriptstyle\frac{1}{3}X+\frac{1}{5}X+\frac{1}{8}X=40</math> | :<math>\scriptstyle\frac{1}{3}X+\frac{1}{5}X+\frac{1}{8}X=40</math> | ||
| − | |style="text-align:right;"|סא | + | |style="text-align:right;"|סא <big>הרי</big> שג' אנשים רצו להתחבר ולשים בחברותה מ' אונקי' זהב<br> |
לאחד יש לו זהב ששוה ג' ליט' האונ‫'<br> | לאחד יש לו זהב ששוה ג' ליט' האונ‫'<br> | ||
והשני יש לו זהב ששוה ה' ליט' האונקי‫'<br> | והשני יש לו זהב ששוה ה' ליט' האונקי‫'<br> | ||
| Line 2,126: | Line 2,512: | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''False Position - common denominator:'''</span> Do it this way, say: [which number has] a third and a fifth and an eighth? Take the common denominator; it is 120. | |
| + | :<math>\scriptstyle{\color{blue}{120}}</math> | ||
|style="text-align:right;"|עשה על זה הדרך אמור שליש וחומש ושמינית אנה ימצאו<br> | |style="text-align:right;"|עשה על זה הדרך אמור שליש וחומש ושמינית אנה ימצאו<br> | ||
בקש המורה והוא ק"כ | בקש המורה והוא ק"כ | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\frac{1}{3}\sdot120=40}}</math> | + | :The third is 40. |
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{3}\sdot120=40}}</math> | ||
|style="text-align:right;"|השלשי הוא מ‫' | |style="text-align:right;"|השלשי הוא מ‫' | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\frac{1}{5}\sdot120=24}}</math> | + | :The fifth is 24. |
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{5}\sdot120=24}}</math> | ||
|style="text-align:right;"|החומש הוא כ"ד | |style="text-align:right;"|החומש הוא כ"ד | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\frac{1}{8}\sdot120=15}}</math> | + | :The eighth is 15. |
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{8}\sdot120=15}}</math> | ||
|style="text-align:right;"|השמינית הוא ט"ו | |style="text-align:right;"|השמינית הוא ט"ו | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Denominator:'''</span> Sum all; they are 79 and this is the denominator. | |
| + | :<math>\scriptstyle{\color{blue}{40+24+15=79}}</math> | ||
|style="text-align:right;"|{{#annot:term|178,1810|5dOg}}צרף כולם{{#annotend:5dOg}} יהיו ע"ט והוא המחלק | |style="text-align:right;"|{{#annot:term|178,1810|5dOg}}צרף כולם{{#annotend:5dOg}} יהיו ע"ט והוא המחלק | ||
|- | |- | ||
| | | | ||
| − | : | + | :If you want to know how much gold the one whose gold is worth 3 liṭra contributes, say: the third is 40. Multiply it by the number of ounces, which is 40 and say: 40 times 40 is 1600. Divide it by 79; the result is 20 and 20 parts of 79. So, he contributed 20 ounces and 20 parts of 79 to the partnership. |
| − | + | :<math>\scriptstyle{\color{blue}{\frac{1}{3}X=\frac{40\sdot40}{79}=\frac{1600}{79}=20+\frac{20}{79}}}</math> | |
|style="text-align:right;"|ואם תרצה לידע כמה זהב ישים אותו שיש לו הזהב ששוה ג' ליט' האונקי‫'<br> | |style="text-align:right;"|ואם תרצה לידע כמה זהב ישים אותו שיש לו הזהב ששוה ג' ליט' האונקי‫'<br> | ||
אמור השלשי הוא מ' ותרבה אותו עם סך האונקי' שהם מ' ואמור מ' פעמי' מ' אלף ות"ר<br> | אמור השלשי הוא מ' ותרבה אותו עם סך האונקי' שהם מ' ואמור מ' פעמי' מ' אלף ות"ר<br> | ||
| Line 2,155: | Line 2,546: | ||
|- | |- | ||
| | | | ||
| − | :::<math>\scriptstyle{\color{blue}{3\sdot20=60}}</math> | + | :If you want to know how much his share is worth, say: 20 ounces yield 60 liṭra. |
| + | :<math>\scriptstyle{\color{blue}{3\sdot20=60}}</math> | ||
|style="text-align:right;"|ואם תרצה לידע כמה שוה חלקו של זה אמור מן הכ' אונקי' יבוא ס' ליט‫' | |style="text-align:right;"|ואם תרצה לידע כמה שוה חלקו של זה אמור מן הכ' אונקי' יבוא ס' ליט‫' | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> for the 20 parts of 79 say: if 79, which is a whole unit, is worth 720 pešuṭim, how much are 20 parts of 79 worth? | |
| + | :<math>\scriptstyle{\color{blue}{1:720=\frac{20}{79}:X}}</math> | ||
|style="text-align:right;"|ומן הכ' חלקי' מע"ט אמור אם ע"ט שהוא אחד שלם שוה תש"כ פש' הכ' חלקי' מע"ט כמה שוים | |style="text-align:right;"|ומן הכ' חלקי' מע"ט אמור אם ע"ט שהוא אחד שלם שוה תש"כ פש' הכ' חלקי' מע"ט כמה שוים | ||
|- | |- | ||
| | | | ||
| − | :::<math>\scriptstyle{\color{blue}{\frac{720\sdot20}{79}=\frac{14400}{79}=182+\frac{22}{79}}}</math> | + | :Say: 20 times 720 is 14400. Divide it by 79; the result is 182 pešuṭim and 22 parts of 79. |
| + | :<math>\scriptstyle{\color{blue}{\frac{720\sdot20}{79}=\frac{14400}{79}=182+\frac{22}{79}}}</math> | ||
|style="text-align:right;"|אמור כ' פעמי' תש"כ י"ד אלפי' ות‫'<br> | |style="text-align:right;"|אמור כ' פעמי' תש"כ י"ד אלפי' ות‫'<br> | ||
| − | חלקם בע"ט יבואו קפ"ב פשוטי' וכ"ב חלקי' מע"ט | + | חלקם ‫<ref>94r</ref>בע"ט יבואו קפ"ב פשוטי' וכ"ב חלקי' מע"ט |
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{X=60+\frac{182+\frac{22}{79}}{20\sdot12}=60+\frac{15}{20}+\frac{2+\frac{22}{79}}{20\sdot12}}}</math> | + | :So, his share is worth 60 liṭra, 15 dinar, 2 pešuṭim and 22 parts of 79. |
| − | + | :<math>\scriptstyle{\color{blue}{X=60+\frac{182+\frac{22}{79}}{20\sdot12}=60+\frac{15}{20}+\frac{2+\frac{22}{79}}{20\sdot12}}}</math> | |
|style="text-align:right;"|הרי ששוה חלקו של זה ס' ליט' וט"ו די' וב"פ וכ"ב חלקי' מע"ט | |style="text-align:right;"|הרי ששוה חלקו של זה ס' ליט' וט"ו די' וב"פ וכ"ב חלקי' מע"ט | ||
|- | |- | ||
| | | | ||
| − | : | + | :Do the same for the others and you will get the answer. |
|style="text-align:right;"|וכן תעשה מן האחרים ויצא לך הענין וכה"ל | |style="text-align:right;"|וכן תעשה מן האחרים ויצא לך הענין וכה"ל | ||
|- | |- | ||
| − | | | + | | |
| + | |||
| + | === <span style=color:green>Give and Take Problem - gatekeeper</span> === | ||
| + | |||
| | | | ||
|- | |- | ||
| | | | ||
| − | :{{#annot: | + | :{{#annot:gatekeeper|627|mqau}}62) A man passed through four towns. |
:In the first town, he doubled all his money and when leaving town the gatekeeper took from him 8 pešuṭim. | :In the first town, he doubled all his money and when leaving town the gatekeeper took from him 8 pešuṭim. | ||
:The same happened in the second and the third towns – in each town he doubled all his money and when leaving town the gatekeeper took from him 8 pešuṭim. | :The same happened in the second and the third towns – in each town he doubled all his money and when leaving town the gatekeeper took from him 8 pešuṭim. | ||
| Line 2,187: | Line 2,584: | ||
:How much money did he have at first? | :How much money did he have at first? | ||
:<math>\scriptstyle2\sdot\left[2\sdot\left[2\sdot\left(2x-8\right)-8\right]-8\right]=8</math> | :<math>\scriptstyle2\sdot\left[2\sdot\left[2\sdot\left(2x-8\right)-8\right]-8\right]=8</math> | ||
| − | |style="text-align:right;"|סב | + | |style="text-align:right;"|סב <big>איש</big> אחד מהלך בד' עיירות<br> |
ובעיר ראשונה הכפיל כל מעותיו ובצאתו מן העיר לקח לו השוער ח"פ<br> | ובעיר ראשונה הכפיל כל מעותיו ובצאתו מן העיר לקח לו השוער ח"פ<br> | ||
וכן בעיר השנית והשלישית הכפיל כל מעותיו ובצאתו מן העיר לקח לו השוער ח"פ בכל עיר<br> | וכן בעיר השנית והשלישית הכפיל כל מעותיו ובצאתו מן העיר לקח לו השוער ח"פ בכל עיר<br> | ||
| − | וכן בעיר הרביעית הכפיל כל מעותיו הנשארים ופרע ח"פ | + | וכן בעיר הרביעית הכפיל כל מעותיו הנשארים ופרע ח"פ ומאז לא נשאר בידו פשוט<br> |
אשאלך כמה מעות היו לו קודם{{#annotend:mqau}} | אשאלך כמה מעות היו לו קודם{{#annotend:mqau}} | ||
|- | |- | ||
| | | | ||
| + | :If you wish to know this, since this man passed through four towns and in each town he paid 8 pešuṭim: | ||
|style="text-align:right;"|אם תרצה לידע אותו עבור כי זה האיש הלך בד' עיירות ובכל עיר פרע ח"פ | |style="text-align:right;"|אם תרצה לידע אותו עבור כי זה האיש הלך בד' עיירות ובכל עיר פרע ח"פ | ||
|- | |- | ||
| | | | ||
| − | : | + | :For the first town, the 8 pešuṭim should be divided into half; they are 4 pešuṭim. |
| + | :<math>\scriptstyle{\color{blue}{\frac{8}{2}=4}}</math> | ||
|style="text-align:right;"|עבור העיר ראשונה צריך אלו הח"פ לחלק לחצי ויהיו ד"פ | |style="text-align:right;"|עבור העיר ראשונה צריך אלו הח"פ לחלק לחצי ויהיו ד"פ | ||
|- | |- | ||
| | | | ||
| − | : | + | :For the second town, the 4 pešuṭim should be divided into half; they are 2 pešuṭim. |
| + | :<math>\scriptstyle{\color{blue}{\frac{4}{2}=2}}</math> | ||
|style="text-align:right;"|ועבו' העיר השנית אלו הד"פ חלק לחצי ויהיו ב"פ | |style="text-align:right;"|ועבו' העיר השנית אלו הד"פ חלק לחצי ויהיו ב"פ | ||
|- | |- | ||
| | | | ||
| − | : | + | :For the third town, the 2 pešuṭim should be divided into half; they are 1 pašuṭ. |
| + | :<math>\scriptstyle{\color{blue}{\frac{2}{2}=1}}</math> | ||
|style="text-align:right;"|ועבו' העיר השלישית אלו הב"פ חלק לחצי ויהיה א"פ | |style="text-align:right;"|ועבו' העיר השלישית אלו הב"פ חלק לחצי ויהיה א"פ | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\frac{1}{2}}}</math> | + | :Divide the pašuṭ into half; it is a half pašuṭ. |
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{2}}}</math> | ||
|style="text-align:right;"|וזה הפשוט חלק לחצי ויהיה חצי פשוט | |style="text-align:right;"|וזה הפשוט חלק לחצי ויהיה חצי פשוט | ||
|- | |- | ||
| | | | ||
| − | : | + | :Then, sum up all together; they are 7 pešuṭim and a half, and this was the original amount of money. |
| + | :<math>\scriptstyle{\color{blue}{x=4+2+1+\frac{1}{2}=7+\frac{1}{2}}}</math> | ||
|style="text-align:right;"|ואסוף אחרי כן כולם יחד ויהיו ז' פשו' וחצי וכך היה הקרן וכה"ל | |style="text-align:right;"|ואסוף אחרי כן כולם יחד ויהיו ז' פשו' וחצי וכך היה הקרן וכה"ל | ||
|- | |- | ||
| − | | | + | | |
| + | |||
| + | === <span style=color:green>Shared Work Problem - Rivers Filling a Fountain</span> === | ||
| + | |||
| | | | ||
|- | |- | ||
| Line 2,227: | Line 2,633: | ||
:If all are flowing together, how long will it take them to fill [the fountain]? | :If all are flowing together, how long will it take them to fill [the fountain]? | ||
:<math>\scriptstyle X+\frac{1}{2}X+\frac{1}{3}X+\frac{1}{4}X=1</math> | :<math>\scriptstyle X+\frac{1}{2}X+\frac{1}{3}X+\frac{1}{4}X=1</math> | ||
| − | |style="text-align:right;"|סג | + | |style="text-align:right;"|סג <big>ד</big> נהרות רצים אל מעיין אחד<br> |
האחד ממלאו ביום אחד<br> | האחד ממלאו ביום אחד<br> | ||
והשני ממלאו בב' ימים<br> | והשני ממלאו בב' ימים<br> | ||
| Line 2,235: | Line 2,641: | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''False Position:'''</span> Do as follows: say: one, a half, a third, and a quarter are found in 12. | |
|style="text-align:right;"|עשה על זה הדרך אמור ראשון וחצי ושליש ורביע ימצא בי"ב | |style="text-align:right;"|עשה על זה הדרך אמור ראשון וחצי ושליש ורביע ימצא בי"ב | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{1\sdot12=12}}</math> | + | :One is 12. |
| + | :<math>\scriptstyle{\color{blue}{1\sdot12=12}}</math> | ||
|style="text-align:right;"|הראשון י"ב | |style="text-align:right;"|הראשון י"ב | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\frac{1}{2}\sdot12=6}}</math> | + | :The half is 6. |
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{2}\sdot12=6}}</math> | ||
|style="text-align:right;"|החצי ו‫' | |style="text-align:right;"|החצי ו‫' | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\frac{1}{3}\sdot12=4}}</math> | + | :The third is 4. |
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{3}\sdot12=4}}</math> | ||
|style="text-align:right;"|השליש ד‫' | |style="text-align:right;"|השליש ד‫' | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\frac{1}{4}\sdot12=3}}</math> | + | :The quarter is 3. |
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{4}\sdot12=3}}</math> | ||
|style="text-align:right;"|הרביע ג‫' | |style="text-align:right;"|הרביע ג‫' | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Denominator:'''</span> Add them together; they are 25 and this is the denominator. | |
| + | :<math>\scriptstyle{\color{blue}{12+6+4+3=25}}</math> | ||
|style="text-align:right;"|חברם יחד הם כ"ה והוא המחלק | |style="text-align:right;"|חברם יחד הם כ"ה והוא המחלק | ||
|- | |- | ||
| | | | ||
| − | : | + | :So, [together] they fill 25 fountains in 12 days: |
|style="text-align:right;"|הרי שבי"ב ימי' ימלאו כ"ה מעינות | |style="text-align:right;"|הרי שבי"ב ימי' ימלאו כ"ה מעינות | ||
|- | |- | ||
| | | | ||
| − | : | + | :The one that fills it in one day - fills 12 fountains in 12 days. |
|style="text-align:right;"|כיצד אותו שממלאהו ביום אחד א"כ בי"ב ימים ימלא י"ב מעינות | |style="text-align:right;"|כיצד אותו שממלאהו ביום אחד א"כ בי"ב ימים ימלא י"ב מעינות | ||
|- | |- | ||
| | | | ||
| − | : | + | :The one that fills it in 2 days - fills 6 fountains in 12 days. |
|style="text-align:right;"|ואותו שממלאהו בב' ימים א"כ בי"ב ימים ימלא ו' מעינות | |style="text-align:right;"|ואותו שממלאהו בב' ימים א"כ בי"ב ימים ימלא ו' מעינות | ||
|- | |- | ||
| | | | ||
| − | : | + | :The one that fills it in 3 days - fills 4 fountains in 12 days. |
|style="text-align:right;"|ואותו שממלאהו בג' ימים א"כ בי"ב ימים ימלא ד' מעיינות | |style="text-align:right;"|ואותו שממלאהו בג' ימים א"כ בי"ב ימים ימלא ד' מעיינות | ||
|- | |- | ||
| | | | ||
| − | : | + | :The one that fills it in 4 days - fills 3 fountains in 12 days. |
|style="text-align:right;"|ואותו שממלאהו בד' ימים א"כ בי"ב ימים ימלא ג' מעיינו‫' | |style="text-align:right;"|ואותו שממלאהו בד' ימים א"כ בי"ב ימים ימלא ג' מעיינו‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | :We find that the four together fill 25 fountains in 12 days. |
|style="text-align:right;"|נמצא שארבעתם יחד ימלאו בי"ב ימים כ"ה מעיינות | |style="text-align:right;"|נמצא שארבעתם יחד ימלאו בי"ב ימים כ"ה מעיינות | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> Say: if 25 fountains are filled in 12 days, how long will it take for one fountain to be filled? | |
| + | :<math>\scriptstyle{\color{blue}{25:12=1:X}}</math> | ||
|style="text-align:right;"|אמור א"כ אם כ"ה מעיינות מתמלאי' בי"ב ימים א' מעיין בכמה מתמלא | |style="text-align:right;"|אמור א"כ אם כ"ה מעיינות מתמלאי' בי"ב ימים א' מעיין בכמה מתמלא | ||
|- | |- | ||
| | | | ||
| − | :: | + | :Say: 1 time 12 is 12. Divide it by 25; the result is 12 parts of 25. So, the fountain is filled in 12 parts of 25 of a day. |
| + | :<math>\scriptstyle{\color{blue}{X=\frac{1\sdot12}{25}=\frac{12}{25}}}</math> | ||
|style="text-align:right;"|אמור א' פעם י"ב הם י"ב<br> | |style="text-align:right;"|אמור א' פעם י"ב הם י"ב<br> | ||
חלקם בכ"ה יבא י"ב חלקי' מכ"ה הרי שבי"ב חלקי' מכ"ה מתמלא ביום המעיין | חלקם בכ"ה יבא י"ב חלקי' מכ"ה הרי שבי"ב חלקי' מכ"ה מתמלא ביום המעיין | ||
|- | |- | ||
| | | | ||
| + | :If you want to know how much of the water flows from each of the rivers to the fountain: | ||
|style="text-align:right;"|ואם תרצה לידע כמה חלק מן המים שם כל אחד מן הנהרות במעיין | |style="text-align:right;"|ואם תרצה לידע כמה חלק מן המים שם כל אחד מן הנהרות במעיין | ||
|- | |- | ||
| | | | ||
| − | : | + | :Say: the first is 12. Say: 12 times 1, for the one that fills it alone in 1 day, is 12. Divide it by 25; the result is 12 parts of 25. |
| + | :<math>\scriptstyle{\color{blue}{X=\frac{1\sdot12}{25}=\frac{12}{25}}}</math> | ||
|style="text-align:right;"|אמור הראשון הוא י"ב אמור י"ב פעמי' א' בעבו' המתמלא שהוא א' הם י"ב חלקם בכ"ה יבוא י"ב חלקי' מכ"ה | |style="text-align:right;"|אמור הראשון הוא י"ב אמור י"ב פעמי' א' בעבו' המתמלא שהוא א' הם י"ב חלקם בכ"ה יבוא י"ב חלקי' מכ"ה | ||
|- | |- | ||
| | | | ||
| − | : | + | :Do this way for the others. |
|style="text-align:right;"|ובזה הדרך תעשה מן האחרים וכה"ל | |style="text-align:right;"|ובזה הדרך תעשה מן האחרים וכה"ל | ||
|- | |- | ||
| | | | ||
| − | : | + | :If you want to know how many fountains they fill in one day, say: 1 time 25 is 25. Divide it by 12; the result is 2 fountains and 1 part of 12. |
| + | :<math>\scriptstyle{\color{blue}{\frac{1\sdot25}{12}=\frac{25}{12}=2+\frac{1}{12}}}</math> | ||
|style="text-align:right;"|ואם תרצה לידע כמה מעיינות ימלאו ביום אחד תאמ' א' פעם כ"ה הם כ"ה חלקם בי"ב יבואו ב' מעיינות וא' חלק מי"ב וכה"ל | |style="text-align:right;"|ואם תרצה לידע כמה מעיינות ימלאו ביום אחד תאמ' א' פעם כ"ה הם כ"ה חלקם בי"ב יבואו ב' מעיינות וא' חלק מי"ב וכה"ל | ||
|- | |- | ||
| − | | | + | | |
| + | |||
| + | === <span style=color:green>Motion Problem – Pursuit</span> === | ||
| + | |||
| | | | ||
|- | |- | ||
| Line 2,315: | Line 2,734: | ||
:<span style=color:red>[See the additional problems below]</span> | :<span style=color:red>[See the additional problems below]</span> | ||
:<math>\scriptstyle10X=\sum_{i=1}^x i</math> | :<math>\scriptstyle10X=\sum_{i=1}^x i</math> | ||
| − | |style="text-align:right;"|סד | + | |style="text-align:right;"|סד <big>הרי</big> אדם אחד שהוא מהלך בכל יום י' מילין<br> |
ואדם אחר מהלך ביום אחד א' מיל וביום שני ב' וביום ג' ג' והוא מוסיף בכל יום מיל אחד<br> | ואדם אחר מהלך ביום אחד א' מיל וביום שני ב' וביום ג' ג' והוא מוסיף בכל יום מיל אחד<br> | ||
בכמה ימים יעמדו בשוה{{#annotend:lHpG}} | בכמה ימים יעמדו בשוה{{#annotend:lHpG}} | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{x=\left(2\sdot10\right)-1=20-1=19}}</math> | + | :Double 10 ; the result is 20. Subtract 1 from 20; it is 19. So, in 19 days they will [walk the same distance]. |
| + | :<math>\scriptstyle{\color{blue}{x=\left(2\sdot10\right)-1=20-1=19}}</math> | ||
|style="text-align:right;"|כפול י' עם י' ויבוא כ' תפחות א' מכ' יהיו י"ט הרי שבי"ט ימים יעמדו בשוה וכה"ל | |style="text-align:right;"|כפול י' עם י' ויבוא כ' תפחות א' מכ' יהיו י"ט הרי שבי"ט ימים יעמדו בשוה וכה"ל | ||
|- | |- | ||
| | | | ||
| − | *If the slower is walking 1, 3, 5, 7, and so on - each day he walks an odd number [of miles] | + | *If the slower is walking 1, 3, 5, 7, and so on - each day he walks an odd number [of miles]. |
:<math>\scriptstyle10X=\sum_{i=1}^x \left(2i-1\right)</math> | :<math>\scriptstyle10X=\sum_{i=1}^x \left(2i-1\right)</math> | ||
|style="text-align:right;"|ואם הצולע מהלך אגה"ז ובכל יום מוסיף והולך פרדים ולא זוגות | |style="text-align:right;"|ואם הצולע מהלך אגה"ז ובכל יום מוסיף והולך פרדים ולא זוגות | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{x=10}}</math> | + | :Take the 10 miles of the one who walks a fixed distance and know that he will catch up with him in 10 days. |
| + | :<math>\scriptstyle{\color{blue}{x=10}}</math> | ||
|style="text-align:right;"|תפוס הי' מילים שמהלך הקבוע ודע שבי' ימים ישיגנו | |style="text-align:right;"|תפוס הי' מילים שמהלך הקבוע ודע שבי' ימים ישיגנו | ||
|- | |- | ||
| Line 2,338: | Line 2,759: | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{x=10-1=9}}</math> | + | :Subtract 1 from the 10 miles of the one who walks a fixed distance and know that he will catch up with him in 9 days. |
| − | |style="text-align:right;"|תפחות א' מן הי' מילין שמהלך הקבוע ואמור כי בט' ישיגנו ימים וכה"ל | + | :<math>\scriptstyle{\color{blue}{x=10-1=9}}</math> |
| + | |style="text-align:right;"|תפחות א' מן הי' מילין שמהלך הקבוע ‫<ref>94v</ref>ואמור כי בט' ישיגנו ימים וכה"ל | ||
|- | |- | ||
| − | | | + | | |
| + | |||
| + | === <span style=color:green>Mixture and Alligation Problem - Goldsmith making rings from several kinds of coins</span> === | ||
| + | |||
| | | | ||
|- | |- | ||
| Line 2,352: | Line 2,777: | ||
:You want to know how many carats per ounce all of them will be together | :You want to know how many carats per ounce all of them will be together | ||
:<math>\scriptstyle\frac{\left(2\sdot14\right)+\left(3\sdot16\right)+\left(5\sdot18\right)}{2+3+5}</math> | :<math>\scriptstyle\frac{\left(2\sdot14\right)+\left(3\sdot16\right)+\left(5\sdot18\right)}{2+3+5}</math> | ||
| − | |style="text-align:right;"|סה | + | |style="text-align:right;"|סה <big>הרי</big> שנתתה לצורף י' אונקיו' זהב לעשות ממנו טבעות<br> |
והב' אונקיו' מהם היה זהב מי"ד קראטי' האונקי‫'<br> | והב' אונקיו' מהם היה זהב מי"ד קראטי' האונקי‫'<br> | ||
והג' אונק' מהם היה זהב מי"ו קרטי' האונק‫'<br> | והג' אונק' מהם היה זהב מי"ו קרטי' האונק‫'<br> | ||
| Line 2,360: | Line 2,785: | ||
|- | |- | ||
| | | | ||
| − | : | + | :Take the amounts of the ounces and the carat. Multiply the carat by its corresponding amount of the ounces. |
| − | |style="text-align:right;"|תפוש סכום האונק' והקרטי' וכפול הקרטי' | + | |style="text-align:right;"|תפוש סכום האונק' והקרטי' וכפול הקרטי' בס<s>כ</s><sup>כ</sup>ום האונק' שלהם |
|- | |- | ||
| | | | ||
| − | : | + | :2 ounces by 14 carat per ounce is 28. |
| + | :<math>\scriptstyle{\color{blue}{2\sdot14=28}}</math> | ||
|style="text-align:right;"|כיצד הב' אונ' מי"ד קרטי' האונ' ת..ם כ"ח | |style="text-align:right;"|כיצד הב' אונ' מי"ד קרטי' האונ' ת..ם כ"ח | ||
|- | |- | ||
| | | | ||
| − | : | + | :3 ounces by 16 carat per ounce is 48. |
| + | :<math>\scriptstyle{\color{blue}{3\sdot16=48}}</math> | ||
|style="text-align:right;"|והג' אונק' מי"ו קרטי' האונ' ת..ם מ"ח | |style="text-align:right;"|והג' אונק' מי"ו קרטי' האונ' ת..ם מ"ח | ||
|- | |- | ||
| | | | ||
| − | + | :five ounces by 18 carat per ounce is 90. | |
| + | :<math>\scriptstyle{\color{blue}{5\sdot18=90}}</math> | ||
|style="text-align:right;"|והה' אונק' מי"ח קרטי' האונ' ת..ם צ‫' | |style="text-align:right;"|והה' אונק' מי"ח קרטי' האונ' ת..ם צ‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | :Add them together; they are 166 carat. |
| + | :<math>\scriptstyle{\color{blue}{28+48+90=166}}</math> | ||
|style="text-align:right;"|חברם יחד הם קס"ו קרטי‫'<br> | |style="text-align:right;"|חברם יחד הם קס"ו קרטי‫'<br> | ||
|- | |- | ||
| | | | ||
| − | : | + | :Divide them into 10 parts, as the number of the ounces; the result is 16 carat and 3-fifths of a carat per ounce. |
| + | :<math>\scriptstyle{\color{blue}{\frac{28+48+90}{2+3+5}=\frac{166}{10}=16+\frac{3}{5}}}</math> | ||
|style="text-align:right;"|חלקם לי' חלקי' כמנין האונ' יבא לכל אונק' י"ו קרטי' וג' חומשי קרטי' וכה"ל | |style="text-align:right;"|חלקם לי' חלקי' כמנין האונ' יבא לכל אונק' י"ו קרטי' וג' חומשי קרטי' וכה"ל | ||
|- | |- | ||
| − | | | + | | |
| + | |||
| + | === <span style=color:green>Divide a Quantity - Price of a Fish Paid by a Group of People</span> === | ||
| + | |||
| | | | ||
|- | |- | ||
| | | | ||
| − | :{{#annot:three men buying a fish|645|4sWF}}66) Three men bought one fish for | + | :{{#annot:three men buying a fish|645|4sWF}}66) Three men bought one fish for 9 pešuṭim. |
:One had a half; the second had a third; and the third had a ninth. | :One had a half; the second had a third; and the third had a ninth. | ||
:You want to know: how much was the share of each in the fish? | :You want to know: how much was the share of each in the fish? | ||
:<math>\scriptstyle\frac{1}{2}X+\frac{1}{3}X+\frac{1}{9}X=9</math> | :<math>\scriptstyle\frac{1}{2}X+\frac{1}{3}X+\frac{1}{9}X=9</math> | ||
| − | |style="text-align:right;"|סו | + | |style="text-align:right;"|סו <big>הרי</big> שג' אנשים קנו א' דג בט"פ<br> |
לאחד יש לו החצי ולשני יש לו השליש ולשלישי יש לו התשיעי<br> | לאחד יש לו החצי ולשני יש לו השליש ולשלישי יש לו התשיעי<br> | ||
ותרצה לידע כמה חלק מן הדג יש לכל אחד{{#annotend:4sWF}} | ותרצה לידע כמה חלק מן הדג יש לכל אחד{{#annotend:4sWF}} | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''False Position:'''</span> Do as follows, say: where are a half, a third, and a ninth found? In 18. | |
|style="text-align:right;"|עשה על זה הדרך אמור אנה ימצא חצי ושליש ותשיעי בי"ח | |style="text-align:right;"|עשה על זה הדרך אמור אנה ימצא חצי ושליש ותשיעי בי"ח | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\frac{1}{2}\sdot18=9}}</math> | + | :The half is 9. |
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{2}\sdot18=9}}</math> | ||
|style="text-align:right;"|החצי הוא ט‫' | |style="text-align:right;"|החצי הוא ט‫' | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\frac{1}{3}\sdot18=6}}</math> | + | :The third is 6. |
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{3}\sdot18=6}}</math> | ||
|style="text-align:right;"|השליש הוא ו‫' | |style="text-align:right;"|השליש הוא ו‫' | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\frac{1}{9}\sdot18=2}}</math> | + | :The ninth is 2. |
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{9}\sdot18=2}}</math> | ||
|style="text-align:right;"|התשיעי הוא ב‫' | |style="text-align:right;"|התשיעי הוא ב‫' | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Denominator:'''</span> Sum them together; they are 17 and this is the denominator. | |
| + | :<math>\scriptstyle{\color{blue}{9+6+2=17}}</math> | ||
|style="text-align:right;"|חברם יחד הם י"ז והוא המחלק | |style="text-align:right;"|חברם יחד הם י"ז והוא המחלק | ||
|- | |- | ||
| | | | ||
| − | : | + | :For the one who has a half of the fish, say: the half is 9. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואותו שיש לו החצי מן הדג אמור החצי הוא ט‫' |
| − | + | |- | |
| + | | | ||
| + | :Multiply it by the fish, which is 1, and say: 9 times 1 is 9. Divide it by 17; his share is 9 parts of 17. | ||
| + | :<math>\scriptstyle{\color{blue}{\frac{1\sdot\left(\frac{1}{2}\sdot18\right)}{17}=\frac{9}{17}}}</math> | ||
| + | |style="text-align:right;"|ותרבה אותו על הדג שהוא א' ואמור ט' פעמי' א' הוא ט‫'<br> | ||
חלקהו בי"ז יבא לחלקו ט' חלקי' מי"ז | חלקהו בי"ז יבא לחלקו ט' חלקי' מי"ז | ||
|- | |- | ||
| | | | ||
| − | : | + | :Do the same for the others and you will get the answer. |
|style="text-align:right;"|וכן תעשה מן האחרים ויצא לך הענין וכה"ל | |style="text-align:right;"|וכן תעשה מן האחרים ויצא לך הענין וכה"ל | ||
|- | |- | ||
| − | | | + | | |
| + | |||
| + | === <span style=color:green>Joint Purchase Problem - If You Give Me - two men - Amounts of Money</span> === | ||
| + | |||
| | | | ||
|- | |- | ||
| | | | ||
| − | :{{#annot:two men, money|664|J6Tf}}67) | + | :{{#annot:two men, money|664|J6Tf}}67) If a man said to his friend: give me 6 pešuṭim and I will have as much as you have. |
| − | :The other one said: if you give me | + | :The other one said: if you give me 6 pešuṭim I will have twice as much as you have. |
| − | :How much money did the first have and how much money did the second have? | + | :I ask you: How much money did the first have and how much money did the second have? |
:<math>\scriptstyle\begin{cases}\scriptstyle X+6=Y-6\\\scriptstyle Y+6=2\sdot\left(X-6\right)\end{cases}</math> | :<math>\scriptstyle\begin{cases}\scriptstyle X+6=Y-6\\\scriptstyle Y+6=2\sdot\left(X-6\right)\end{cases}</math> | ||
| − | |style="text-align:right;"|סז | + | |style="text-align:right;"|סז <big>אם</big> יאמר אדם לחבירו תן לי ו"פ יהיה לי כאשר יש לך<br> |
והאחר אמ' אם תתן לי ו"פ יהיה לי פי שנים ממך<br> | והאחר אמ' אם תתן לי ו"פ יהיה לי פי שנים ממך<br> | ||
אשאלך כמה מעות היו לאחד וכמה מעות היו לאחר{{#annotend:J6Tf}} | אשאלך כמה מעות היו לאחד וכמה מעות היו לאחר{{#annotend:J6Tf}} | ||
|- | |- | ||
| | | | ||
| − | ::the one who | + | :Say: 6 times 7 is 42 and this is the amount of money of the one who said: give me 6 pešuṭim and I will have twice as much as you have. |
| + | :<math>\scriptstyle{\color{blue}{Y=6\sdot7=42}}</math> | ||
|style="text-align:right;"|תאמ' ו' פעמי' ז' מ"ב וכך מעות היו לאיש שאמר תן לי ו"פ יהיה לי כפול ממך | |style="text-align:right;"|תאמ' ו' פעמי' ז' מ"ב וכך מעות היו לאיש שאמר תן לי ו"פ יהיה לי כפול ממך | ||
|- | |- | ||
| | | | ||
| − | :: | + | :For the amount of money of the other, say: 5 times 6 is 30 and this is what he has. |
| + | :<math>\scriptstyle{\color{blue}{X=5\sdot6=30}}</math> | ||
|style="text-align:right;"|ולמעות האחר תאמ' ה' פעמ' ו' ל' וכן היו לו כה"ל | |style="text-align:right;"|ולמעות האחר תאמ' ה' פעמ' ו' ל' וכן היו לו כה"ל | ||
|- | |- | ||
| − | | | + | | |
| + | |||
| + | === <span style=color:green>Interest and Discount Problem - find the fund</span> === | ||
| + | |||
| | | | ||
|- | |- | ||
| | | | ||
| − | :{{#annot:no time|666|f5jn}}68) I produced two pešuṭim from one pašuṭ, I produced three [pešuṭim] from two [pešuṭim], four from three, five from four, and six from five, and I had a total of 400 pešuṭim. | + | :{{#annot:no time|666|f5jn}}68) If you are told: I produced two pešuṭim from one pašuṭ, I produced three [pešuṭim] from two [pešuṭim], four from three, five from four, and six from five, and I had a total of 400 pešuṭim. |
| − | :How much was the money invested? | + | :I ask you: How much was the money invested? |
:<math>\scriptstyle{\color{blue}{\frac{6}{1}=\frac{400}{X}}}</math> | :<math>\scriptstyle{\color{blue}{\frac{6}{1}=\frac{400}{X}}}</math> | ||
| − | |style="text-align:right;"|סח | + | |style="text-align:right;"|סח <big>אם</big> נאמר לך אני פלני עשיתי מא"פ ב' ומב' עשיתי ג' ומג' ד' ומד' ה' ומה' ו' והיו לי בין קרן וריוח ת"פ<br> |
אשאלך כמה היה הקרן{{#annotend:f5jn}} | אשאלך כמה היה הקרן{{#annotend:f5jn}} | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> Do as follows: you produced 2 from one, 3 from 2, 4 from 3, 5 from 4, and 6 from 5; so you can see that 6 are produced from 1. How much will yield 400 pešuṭim? | |
| + | :<math>\scriptstyle{\color{blue}{6:1=400:X}}</math> | ||
|style="text-align:right;"|עשה כן עת' כי מאחד עשית ב' ומב' ג' ומג' ד' ומד' ה' ומה' ו‫'<br> | |style="text-align:right;"|עשה כן עת' כי מאחד עשית ב' ומב' ג' ומג' ד' ומד' ה' ומה' ו‫'<br> | ||
א"כ צריך לראות כי ו' יצאו מא' א"כ מאיין מצים לצאת ת"פ | א"כ צריך לראות כי ו' יצאו מא' א"כ מאיין מצים לצאת ת"פ | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{x=\frac{1\sdot400}{6}=66+\frac{2}{3}}}</math> | + | :Do like this: 1 time 400 pešuṭim; divide them by 6; they are 66 pešuṭim and 2-thirds of one pašuṭ and this is the investment. |
| + | :<math>\scriptstyle{\color{blue}{x=\frac{1\sdot400}{6}=66+\frac{2}{3}}}</math> | ||
|style="text-align:right;"|עשה כן א' פעם ת"פ וחלקם בו' יהיו ס"ו פשו' וב' שלישי פשוט וכן היה הקרן | |style="text-align:right;"|עשה כן א' פעם ת"פ וחלקם בו' יהיו ס"ו פשו' וב' שלישי פשוט וכן היה הקרן | ||
|- | |- | ||
| − | | | + | | |
| + | |||
| + | === <span style=color:green>Exchange Problems</span> === | ||
| + | |||
| | | | ||
|- | |- | ||
| | | | ||
| − | :{{#annot:two currencies|632|frHK}}69) 20 dinar of Ancona are worth | + | :{{#annot:two currencies|632|frHK}}69) 20 dinar of Ancona are worth 31 pešuṭim and a half of Bologna. |
| − | :How many [of Bologna] are | + | :How many [of Bologna] are 37 dinar and a half of Ancona worth? |
:<math>\scriptstyle\frac{20}{31+\frac{1}{2}}=\frac{37+\frac{1}{2}}{X}</math> | :<math>\scriptstyle\frac{20}{31+\frac{1}{2}}=\frac{37+\frac{1}{2}}{X}</math> | ||
| − | |style="text-align:right;"|סט | + | |style="text-align:right;"|סט <big>כ</big> די' אונקוניטני לחילוף בולייניני' לא"פ וחצי הליט' שוה פחות מן הבולונייני‫'<br> |
כמה יבא לל"ז די' וחצי מאנקונטני' מחלוף{{#annotend:frHK}} | כמה יבא לל"ז די' וחצי מאנקונטני' מחלוף{{#annotend:frHK}} | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{37\sdot31=1147}}</math> | + | :Do as follows: 37 times 31 is 1147. |
| + | :<math>\scriptstyle{\color{blue}{37\sdot31=1147}}</math> | ||
|style="text-align:right;"|עשה כן ל"ז פעמי' ל"א הם אלף וקמ"ז | |style="text-align:right;"|עשה כן ל"ז פעמי' ל"א הם אלף וקמ"ז | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\frac{1}{2}\sdot31=15+\frac{1}{2}}}</math> | + | :Then, say: how much is a half of 31? It is 15 and a half. |
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{2}\sdot31=15+\frac{1}{2}}}</math> | ||
|style="text-align:right;"|ואחר אמור איזהו החצי מל"א הוא ט"ו וחצי | |style="text-align:right;"|ואחר אמור איזהו החצי מל"א הוא ט"ו וחצי | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\frac{1}{2}\sdot37=18+\frac{1}{2}}}</math> | + | :How much is a half of 37? It is 18 and a half. |
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{2}\sdot37=18+\frac{1}{2}}}</math> | ||
|style="text-align:right;"|ומהו החצי מל"ז והוא י"ח וחצי | |style="text-align:right;"|ומהו החצי מל"ז והוא י"ח וחצי | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\frac{1}{2}\sdot\frac{1}{2}=\frac{1}{4}}}</math> | + | :How much is a half of a half? It is a quarter. |
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{2}\sdot\frac{1}{2}=\frac{1}{4}}}</math> | ||
|style="text-align:right;"|ומהו החצי מן החצי הוא רביע | |style="text-align:right;"|ומהו החצי מן החצי הוא רביע | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> Sum [1147], 15 and a half, 18 and a half, and a quarter; they are 1181 and a quarter. Divide them into 20 parts; the result is 59 pešuṭim, 1 part of 20 and 1 part of 80, i.e. 5 parts of 80. | |
| − | |||
|style="text-align:right;"|ואסוף ט"ו וחצי וי"ח וחצי ורביע יהיו אלף וקפ"א ורביע<br> | |style="text-align:right;"|ואסוף ט"ו וחצי וי"ח וחצי ורביע יהיו אלף וקפ"א ורביע<br> | ||
חלק אותם לכ' חלקי' יבא נ"ט פשו' וא' חלק מכ' וא' חלק מפ‫'<br> | חלק אותם לכ' חלקי' יבא נ"ט פשו' וא' חלק מכ' וא' חלק מפ‫'<br> | ||
דהיינו ה' חלקי' מפ' וכה"ל | דהיינו ה' חלקי' מפ' וכה"ל | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | :<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle x&\scriptstyle=\frac{\left(37+\frac{1}{2}\right)\sdot\left(31+\frac{1}{2}\right)}{20}=\frac{1147+\left(15+\frac{1}{2}\right)+\left(18+\frac{1}{2}\right)+\frac{1}{4}}{20}=\frac{1181+\frac{1}{4}}{20}\\&\scriptstyle=59+\frac{1}{20}+\frac{1}{80}=59+\frac{5}{80}\\\end{align}}}</math> | ||
|- | |- | ||
| | | | ||
| − | *How many [of Bologna] are | + | *If it is said: How many [of Bologna] are 91 dinar and a quarter of Ancona worth? |
:<math>\scriptstyle\frac{20}{31+\frac{1}{2}}=\frac{91+\frac{1}{4}}{X}</math> | :<math>\scriptstyle\frac{20}{31+\frac{1}{2}}=\frac{91+\frac{1}{4}}{X}</math> | ||
|style="text-align:right;"|ואם יאמר לצ"א די' ורביע כמה יבא כמו כן | |style="text-align:right;"|ואם יאמר לצ"א די' ורביע כמה יבא כמו כן | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{91\sdot31=2821}}</math> | + | :Multiply 91 times 31; it is 2821. |
| + | :<math>\scriptstyle{\color{blue}{91\sdot31=2821}}</math> | ||
|style="text-align:right;"|תרבה צ"א פעמי' ל"א והם אלפיים ותתכ"א | |style="text-align:right;"|תרבה צ"א פעמי' ל"א והם אלפיים ותתכ"א | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\frac{1}{4}\sdot31=7+\frac{3}{4}}}</math> | + | :Then, say: how much is a quarter of 31? It is 7 and 3-quarters. |
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{4}\sdot31=7+\frac{3}{4}}}</math> | ||
|style="text-align:right;"|ואחר אמור איזהו הרביע מל"א הוא ז' וג' רבעי‫' | |style="text-align:right;"|ואחר אמור איזהו הרביע מל"א הוא ז' וג' רבעי‫' | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\frac{1}{2}\sdot91=45+\frac{1}{2}}}</math> | + | :How much is a half of 91? It is 45 and a half. |
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{2}\sdot91=45+\frac{1}{2}}}</math> | ||
|style="text-align:right;"|ומה הוא החצי מצ"א והוא מ"ה וחצי | |style="text-align:right;"|ומה הוא החצי מצ"א והוא מ"ה וחצי | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\frac{1}{2}\sdot\frac{1}{4}=\frac{1}{8}}}</math> | + | :How much is a half of a quarter? It is an eighth. |
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{2}\sdot\frac{1}{4}=\frac{1}{8}}}</math> | ||
|style="text-align:right;"|ומהו החצי מן הרביע הוא שמינית | |style="text-align:right;"|ומהו החצי מן הרביע הוא שמינית | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> Sum them with 2821; they are 2874, a quarter and an eighth. Divide them into 20 parts; they are 143 pešuṭim, 3-quarters and 1 part of 20, i.e. 144 pešuṭim minus a fifth. | |
| − | |||
|style="text-align:right;"|ו{{#annot:term|178,1190|0AVj}}אסוף עם{{#annotend:0AVj}} אלפים ותתכ"א ויהיו אלפי' ותתע"ד וא' רביע וא' שמינית<br> | |style="text-align:right;"|ו{{#annot:term|178,1190|0AVj}}אסוף עם{{#annotend:0AVj}} אלפים ותתכ"א ויהיו אלפי' ותתע"ד וא' רביע וא' שמינית<br> | ||
| − | וחלקם בכ' חלקי' ויהיו קמ"ג פשו' וג' רבעים וא' חלק מכ‫'<br> | + | וחלקם בכ' חלקי' ויהיו ‫<ref>95r</ref>קמ"ג פשו' וג' רבעים וא' חלק מכ‫'<br> |
דהיינו קמ"ד פשו' פחות חומש וכה"ל | דהיינו קמ"ד פשו' פחות חומש וכה"ל | ||
|- | |- | ||
| − | | | + | | colspan="2"| |
| + | :<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle x&\scriptstyle=\frac{\left(91+\frac{1}{4}\right)\sdot\left(31+\frac{1}{2}\right)}{20}=\frac{2821+\left(7+\frac{3}{4}\right)+\left(45+\frac{1}{2}\right)+\frac{1}{8}}{20}=\frac{2874+\frac{1}{4}+\frac{1}{8}}{20}\\&\scriptstyle\approx143+\frac{3}{4}+\frac{1}{20}=144-\frac{1}{5}\\\end{align}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | === <span style=color:green>Interest and Discount Problem</span> === | ||
| + | |||
| | | | ||
|- | |- | ||
| Line 2,531: | Line 3,000: | ||
:{{#annot:a year|666|qKw0}}70) A certain amount produced 17 liṭra in one year at 3 pešuṭim for one liṭra a month. | :{{#annot:a year|666|qKw0}}70) A certain amount produced 17 liṭra in one year at 3 pešuṭim for one liṭra a month. | ||
:How much was the money invested? | :How much was the money invested? | ||
| − | :<math>\scriptstyle X+\left( | + | :<math>\scriptstyle X+\left(X\sdot\frac{3}{20}\right)=17</math> |
| − | |style="text-align:right;"|ע | + | |style="text-align:right;"|ע <big>חשבון</big> אחר שבין קרן וריוח היו בשנה אחת י"ז ליט' לחשבון גפה"ה<br> |
כמה היה הקרן{{#annotend:qKw0}} | כמה היה הקרן{{#annotend:qKw0}} | ||
|- | |- | ||
| | | | ||
| + | :Calculate and say: 23 liṭra at 3 pešuṭim for one liṭra a month are 20 liṭra of the fund and 3 liṭra of interest at the end of the year. | ||
|style="text-align:right;"|תפוס חשבון אחד ואמור כ"ג ליט' לחשבון גפה"ה היו בסוף השנה כ' ליט' מקרן וג' ליט' מריוח | |style="text-align:right;"|תפוס חשבון אחד ואמור כ"ג ליט' לחשבון גפה"ה היו בסוף השנה כ' ליט' מקרן וג' ליט' מריוח | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> Say: if 23 is 20, how much is 17? | |
| + | :<math>\scriptstyle{\color{blue}{23:20=17:X}}</math> | ||
|style="text-align:right;"|ואמור אם כ"ג היה כ' י"ז כמה היה | |style="text-align:right;"|ואמור אם כ"ג היה כ' י"ז כמה היה | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{x=\frac{17\sdot20}{23}=\frac{340}{23}=14+\frac{15}{20}+\frac{\frac{15}{23}}{20}}}</math> | + | :Say: 17 times 20 is 340. Divide it by 23; it is 14 liṭra, plus 15 dinar and 15 parts of 23 of a [dinar]. |
| − | + | :<math>\scriptstyle{\color{blue}{x=\frac{17\sdot20}{23}=\frac{340}{23}=14+\frac{15}{20}+\frac{\frac{15}{23}}{20}}}</math> | |
|style="text-align:right;"|אמור י"ז פעמי' כ' ש"מ<br> | |style="text-align:right;"|אמור י"ז פעמי' כ' ש"מ<br> | ||
חלקם על כ"ג יהיו י"ד ליט' וט"ו די' נוס' וט"ו חלקי' מכ"ג בפשוט וכה"ל | חלקם על כ"ג יהיו י"ד ליט' וט"ו די' נוס' וט"ו חלקי' מכ"ג בפשוט וכה"ל | ||
|- | |- | ||
| − | | | + | | |
| + | |||
| + | === <span style=color:green>Pricing Problem - Find the Amount</span> === | ||
| + | |||
| | | | ||
|- | |- | ||
| | | | ||
| − | :{{#annot:leather|629|S3ri}}71) One kikkar of leather for | + | :{{#annot:leather|629|S3ri}}71) If you are asked: One kikkar of leather for 21 liṭra and a half. |
| − | :How much leather [can be bought] for | + | :How much leather [can be bought] for 12 liṭra and a quarter? |
:<math>\scriptstyle\frac{100}{21+\frac{1}{2}}=\frac{X}{12+\frac{1}{4}}</math> | :<math>\scriptstyle\frac{100}{21+\frac{1}{2}}=\frac{X}{12+\frac{1}{4}}</math> | ||
| − | |style="text-align:right;"|עא | + | |style="text-align:right;"|עא <big>אם</big> נשאלת בכ"א ליט' וחצי הככר מן העורות עבור י"ב ליט' ורביע כמה עורות יבא{{#annotend:S3ri}} |
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''False Position - denominator:'''</span> Add 21 and a half with 21 and a half; they are 43 and this is the denominator. | |
| + | :<math>\scriptstyle{\color{blue}{\left(21+\frac{1}{2}\right)+\left(21+\frac{1}{2}\right)=43}}</math> | ||
|style="text-align:right;"|עשה כ"א וחצי וכ"א וחצי ויהיו מ"ג וזהו המחלק | |style="text-align:right;"|עשה כ"א וחצי וכ"א וחצי ויהיו מ"ג וזהו המחלק | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{2\sdot100=200}}</math> | + | :Then, multiply 2 times 100; they are two hundred. |
| + | :<math>\scriptstyle{\color{blue}{2\sdot100=200}}</math> | ||
|style="text-align:right;"|ואחר עשה ב' פעמי' ק' והם מאתיי‫' | |style="text-align:right;"|ואחר עשה ב' פעמי' ק' והם מאתיי‫' | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> Multiply 12 liṭra and a quarter by two hundred; they are 2450. Divide into 43 parts; they are 56 leathers and 42 parts of 43 of one unit. | |
| + | :<math>\scriptstyle{\color{blue}{x=\frac{200\sdot\left(12+\frac{1}{4}\right)}{43}=\frac{2450}{43}=56+\frac{42}{43}}}</math> | ||
|style="text-align:right;"|ותרבה י"ב ליט' ורביע עם מאתיים ויהיו אלפיי' ות"נ<br> | |style="text-align:right;"|ותרבה י"ב ליט' ורביע עם מאתיים ויהיו אלפיי' ות"נ<br> | ||
וחלק במ"ג חלקים ויהיו נ"ו עורות ומ"ב חלקי' ממ"ג מא' עור וכה"ל | וחלק במ"ג חלקים ויהיו נ"ו עורות ומ"ב חלקי' ממ"ג מא' עור וכה"ל | ||
|- | |- | ||
| | | | ||
| − | *How much leather [can be bought] for | + | *If it is said: How much leather [can be bought] for 71 liṭra and a half? |
:<math>\scriptstyle\frac{100}{21+\frac{1}{2}}=\frac{X}{71+\frac{1}{2}}</math> | :<math>\scriptstyle\frac{100}{21+\frac{1}{2}}=\frac{X}{71+\frac{1}{2}}</math> | ||
|style="text-align:right;"|ואם יאמר בע"א ליט' וחצי כמה עורות יבואו לו כמו כן | |style="text-align:right;"|ואם יאמר בע"א ליט' וחצי כמה עורות יבואו לו כמו כן | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> Multiply 71 liṭra and a half by 200; they are 143 kikkar. Divide by 43; they are 332 leathers and 24 parts of 43 of one unit. | |
| − | + | :<math>\scriptstyle{\color{blue}{x=\frac{100\sdot2\sdot\left(71+\frac{1}{2}\right)}{\left(21+\frac{1}{2}\right)+\left(21+\frac{1}{2}\right)}=\frac{100\sdot143}{43}=332+\frac{24}{43}}}</math> | |
| − | |style="text-align:right;"|תרבה ע"א ליט' וחצי עם | + | |style="text-align:right;"|תרבה ע"א ליט' וחצי עם ר' ויהיו קמ"ג ככרים<br> |
וחלק במ"ג ויהיו של"ב עורות וכ"ד חלקי' ממ"ג מא' עור וכה"ל | וחלק במ"ג ויהיו של"ב עורות וכ"ד חלקי' ממ"ג מא' עור וכה"ל | ||
|- | |- | ||
| − | | | + | | |
| + | |||
| + | === <span style=color:green>Divide a Quantity - Sharing Food</span> === | ||
| + | |||
| | | | ||
|- | |- | ||
| Line 2,591: | Line 3,071: | ||
:How should they share the [five pešiṭim]? | :How should they share the [five pešiṭim]? | ||
:<math>\scriptstyle\left[\left(2-\frac{5}{3}\right)\sdot X\right]+\left[\left(3-\frac{5}{3}\right)\sdot X\right]=5</math> | :<math>\scriptstyle\left[\left(2-\frac{5}{3}\right)\sdot X\right]+\left[\left(3-\frac{5}{3}\right)\sdot X\right]=5</math> | ||
| − | |style="text-align:right;"|עב | + | |style="text-align:right;"|עב <big>ב</big> אנשים היו יושבים לאכול<br> |
לאחד יש לו ב' לחמים ולשני ג‫'<br> | לאחד יש לו ב' לחמים ולשני ג‫'<br> | ||
בא השלישי והיסב לאכול עמהם ואכלו כן שלשתם אלו הה' לחמים<br> | בא השלישי והיסב לאכול עמהם ואכלו כן שלשתם אלו הה' לחמים<br> | ||
| − | לאחר שאכלו ושתו נתן השלשי שבא לאכול עמהם | + | לאחר שאכלו ושתו נתן השלשי שבא לאכול עמהם ה"פ לאותם השנים<br> |
היאך יחלקום{{#annotend:Rh1d}} | היאך יחלקום{{#annotend:Rh1d}} | ||
|- | |- | ||
| | | | ||
| − | : | + | :Say how many pieces of bread each ate: 1 loaf of bread and 2-thirds. |
| + | :<math>\scriptstyle{\color{blue}{\frac{5}{3}=1+\frac{2}{3}}}</math> | ||
|style="text-align:right;"|אמור כמה חלקי' מן הלחם אכל כל אחד ואחד א' לחם וב' שלישיות | |style="text-align:right;"|אמור כמה חלקי' מן הלחם אכל כל אחד ואחד א' לחם וב' שלישיות | ||
|- | |- | ||
| | | | ||
| − | : | + | :So, the one who had 2 loaves of bread and ate 1 loaf of bread and 2-thirds lost one third of a loaf. |
| + | :<math>\scriptstyle{\color{blue}{2-\left(1+\frac{2}{3}\right)=\frac{1}{3}}}</math> | ||
|style="text-align:right;"|א"כ אותו שהיו לו ב' לחמים ואכל א' לחם וב' שלשיות לא הפסיד כי אם שליש לחם | |style="text-align:right;"|א"כ אותו שהיו לו ב' לחמים ואכל א' לחם וב' שלשיות לא הפסיד כי אם שליש לחם | ||
|- | |- | ||
| | | | ||
| − | : | + | :The one who had 3 loaves of bread and ate 1 loaf of bread and 2-thirds lost one loaf of bread and a third, i.e. 4-thirds. |
| + | :<math>\scriptstyle{\color{blue}{3-\left(1+\frac{2}{3}\right)=1+\frac{1}{3}=\frac{4}{3}}}</math> | ||
|style="text-align:right;"|ואותו שהיו לו ג' לחמים ואכל א' לחם וב' שלשיות הפסיד א' לחם ושליש דהיינו ד' שלשיות | |style="text-align:right;"|ואותו שהיו לו ג' לחמים ואכל א' לחם וב' שלשיות הפסיד א' לחם ושליש דהיינו ד' שלשיות | ||
|- | |- | ||
| | | | ||
| − | : | + | :Therefore, the one who had 2 loaves of bread will receive 1 pašuṭ. |
| + | :<math>\scriptstyle{\color{blue}{a_2=1}}</math> | ||
|style="text-align:right;"|א"כ אותו שהיו לו ב' לחמי' יקבל א"פ | |style="text-align:right;"|א"כ אותו שהיו לו ב' לחמי' יקבל א"פ | ||
|- | |- | ||
| | | | ||
| − | : | + | :And the other will receive 4 pešiṭim. |
| + | :<math>\scriptstyle{\color{blue}{a_3=4}}</math> | ||
|style="text-align:right;"|והאחר יקבל ד"פ | |style="text-align:right;"|והאחר יקבל ד"פ | ||
|- | |- | ||
| − | |||
| | | | ||
| − | |- | + | |
| − | | | + | === <span style=color:green>Find a Number Problems - Multiplication of fractions</span> === |
| + | |||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | ==== <span style=color:green>Fractions by Fractions</span> ==== | ||
| + | |||
| | | | ||
|- | |- | ||
| | | | ||
| − | :{{#annot:⅘×⅘|17|kvFj}}73) How much are four fifths by four fifths? | + | :{{#annot:⅘×⅘|17|kvFj}}73) If a man asks you: How much are four fifths by four fifths? |
:<math>\scriptstyle\frac{4}{5}\times\frac{4}{5}</math> | :<math>\scriptstyle\frac{4}{5}\times\frac{4}{5}</math> | ||
| − | |style="text-align:right;"|עג | + | |style="text-align:right;"|עג <big>אם</big> ישאלך אדם כמה הוא ד' חמשיות על ד' חמשיות{{#annotend:kvFj}} |
|- | |- | ||
| | | | ||
| − | + | :{{#annot:⅕×⅕|17|ubzN}}Say: if he had asked for one fifth by one fifth. | |
| + | :<math>\scriptstyle\frac{1}{5}\times\frac{1}{5}</math> | ||
|style="text-align:right;"|תאמר אם היה שואל א' חמישית על א' חמישית{{#annotend:ubzN}} | |style="text-align:right;"|תאמר אם היה שואל א' חמישית על א' חמישית{{#annotend:ubzN}} | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\frac{1}{5}\times\frac{1}{5}=\frac{1}{5\sdot5}=\frac{1}{25}}}</math> | + | :You would have said: 5 times 5 is 25, so the result would have been one part of 25. |
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{5}\times\frac{1}{5}=\frac{1}{5\sdot5}=\frac{1}{25}}}</math> | ||
|style="text-align:right;"|הייתה אומר ה' פעמי' ה' כ"ה והיה עולה א' חלק מכ"ה | |style="text-align:right;"|הייתה אומר ה' פעמי' ה' כ"ה והיה עולה א' חלק מכ"ה | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\frac{4}{5}\times\frac{4}{5}=\frac{4\sdot4}{25}=\frac{16}{25}}}</math> | + | :Now that he asks for 4-fifths by 4-fifths, you say: 4 times 4 is 16. We find that the result is 16 parts of 25. |
| + | :<math>\scriptstyle{\color{blue}{\frac{4}{5}\times\frac{4}{5}=\frac{4\sdot4}{25}=\frac{16}{25}}}</math> | ||
|style="text-align:right;"|ועתה שהוא שואל ד' חמשיות על ד' חמשיות עדיין תאמ' ד' פעמי' ד' י"ו נמצא שיבא י"ו חלקי' מכ"ה | |style="text-align:right;"|ועתה שהוא שואל ד' חמשיות על ד' חמשיות עדיין תאמ' ד' פעמי' ד' י"ו נמצא שיבא י"ו חלקי' מכ"ה | ||
|- | |- | ||
| − | |||
| | | | ||
| − | + | :{{#annot:⁷/₉×⁴/₇|17|S123}}74) If a man asks you: How much are seven ninths by four sevenths? | |
| − | |||
| − | :{{#annot:⁷/₉×⁴/₇|17|S123}}74) How much are seven ninths by four sevenths? | ||
:<math>\scriptstyle\frac{7}{9}\times\frac{4}{7}</math> | :<math>\scriptstyle\frac{7}{9}\times\frac{4}{7}</math> | ||
| − | |style="text-align:right;"|עד | + | |style="text-align:right;"|עד <big>אי"א</big> כמה הוא ז' תשיעיות על ד' שבעיות{{#annotend:S123}} |
|- | |- | ||
| | | | ||
| − | + | :{{#annot:⅑×⅐|17|P04R}}If he had asked for one ninth by one seventh. | |
| + | :<math>\scriptstyle\frac{1}{9}\times\frac{1}{7}</math> | ||
|style="text-align:right;"|תאמ' אם היה שואל א' תשיעית על א' שבעית{{#annotend:P04R}} | |style="text-align:right;"|תאמ' אם היה שואל א' תשיעית על א' שבעית{{#annotend:P04R}} | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\frac{1}{9}\times\frac{1}{7}=\frac{1}{7\sdot9}=\frac{1}{63}}}</math> | + | :You would have said: 7 times 9 is 63. We find that the result would have been one part of 63. |
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{9}\times\frac{1}{7}=\frac{1}{7\sdot9}=\frac{1}{63}}}</math> | ||
|style="text-align:right;"|הייתה אומ' ז' פעמ' ט' הם ס"ג נמצא שהיה עולה א' חלק מס"ג | |style="text-align:right;"|הייתה אומ' ז' פעמ' ט' הם ס"ג נמצא שהיה עולה א' חלק מס"ג | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\frac{7}{9}\times\frac{4}{7}=\frac{7\sdot4}{63}=\frac{28}{63}}}</math> | + | :Now that he asks for 7-ninths by 4-sevenths, you say: 7 times 4 is 28. We find that the result is 28 parts of 63. |
| + | :<math>\scriptstyle{\color{blue}{\frac{7}{9}\times\frac{4}{7}=\frac{7\sdot4}{63}=\frac{28}{63}}}</math> | ||
|style="text-align:right;"|ועתה שהוא שואל ז' תשעיות על ד' שבעיות עדיין תאמ' ז' פעמ' ד' כ"ח נמצא שיבא כ"ח חלקי' מס"ג וכה"ל | |style="text-align:right;"|ועתה שהוא שואל ז' תשעיות על ד' שבעיות עדיין תאמ' ז' פעמ' ד' כ"ח נמצא שיבא כ"ח חלקי' מס"ג וכה"ל | ||
|- | |- | ||
| − | | | + | | |
| + | ==== <span style=color:green>Integers and Fractions</span> ==== | ||
| + | |||
| | | | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:3⅖×2⁴/₇|156|9pSN}}How much are three integers and two fifths by two integers and four sevenths? | + | *{{#annot:3⅖×2⁴/₇|156|9pSN}}If a man asks you: How much are three integers and two fifths by two integers and four sevenths? |
:<math>\scriptstyle\left(3+\frac{2}{5}\right)\times\left(2+\frac{4}{7}\right)</math> | :<math>\scriptstyle\left(3+\frac{2}{5}\right)\times\left(2+\frac{4}{7}\right)</math> | ||
| − | |style="text-align:right;"|אי"א ג' שלמים וב' חמשיות על ב' שלמים וד' שבעיות כמה הוא{{#annotend:9pSN}} | + | |style="text-align:right;"|<big>אי"א</big> ג' שלמים וב' חמשיות על ב' שלמים וד' שבעיות כמה הוא{{#annotend:9pSN}} |
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{3\sdot2=6}}</math> | + | :Say: 3 times 2 is 6 integers. |
| + | :<math>\scriptstyle{\color{blue}{3\sdot2=6}}</math> | ||
|style="text-align:right;"|תאמ' ג' פעמ' ב' הם ו' שלמים | |style="text-align:right;"|תאמ' ג' פעמ' ב' הם ו' שלמים | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{3\sdot\frac{4}{7}=\frac{12}{7}=1+\frac{5}{7}=1+\frac{25}{35}}}</math> | + | :Say: 3 times 4-sevenths are 12 that are one integer and 5-sevenths, which is 25 parts of 35. |
| + | :<math>\scriptstyle{\color{blue}{3\sdot\frac{4}{7}=\frac{12}{7}=1+\frac{5}{7}=1+\frac{25}{35}}}</math> | ||
|style="text-align:right;"|אח"כ תאמר ג' פעמ' ד' שבעיות הם י"ב שהוא אחד שלם וה' שבעיות שהוא כ"ה חלקי' מל"ה | |style="text-align:right;"|אח"כ תאמר ג' פעמ' ד' שבעיות הם י"ב שהוא אחד שלם וה' שבעיות שהוא כ"ה חלקי' מל"ה | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{2\sdot\frac{2}{5}=\frac{4}{5}=\frac{28}{35}}}</math> | + | :Say: 2 times 2-fifths are 4-fifths, which is 28 parts of 35. |
| + | :<math>\scriptstyle{\color{blue}{2\sdot\frac{2}{5}=\frac{4}{5}=\frac{28}{35}}}</math> | ||
|style="text-align:right;"|אח"כ תאמ' ב' פעמ' ב' חמשיות הם ד' חמשיות שהם כ"ח חלקי' מל"ה | |style="text-align:right;"|אח"כ תאמ' ב' פעמ' ב' חמשיות הם ד' חמשיות שהם כ"ח חלקי' מל"ה | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\frac{2}{5}\sdot\frac{4}{7}=\frac{8}{35}}}</math> | + | :Say: 2-fifths by 4-sevenths is 8 parts of 35. |
| + | :<math>\scriptstyle{\color{blue}{\frac{2}{5}\sdot\frac{4}{7}=\frac{8}{35}}}</math> | ||
|style="text-align:right;"|ואחרי כן תאמ' ב' חמשיות על ד' שבעיות הוא ח' חלקי' מל"ה | |style="text-align:right;"|ואחרי כן תאמ' ב' חמשיות על ד' שבעיות הוא ח' חלקי' מל"ה | ||
|- | |- | ||
| | | | ||
| − | : | + | :Add them up; the result is 8 integers and 26 parts of 35. |
|style="text-align:right;"|צרף כלם יחד יבא ח' שלמים וכ"ו חלקי' מל"ה וכן יבא וכה"ל | |style="text-align:right;"|צרף כלם יחד יבא ח' שלמים וכ"ו חלקי' מל"ה וכן יבא וכה"ל | ||
|- | |- | ||
| − | | | + | | colspan="2"| |
| − | + | :<math>\scriptstyle{\color{blue}{\left(3+\frac{2}{5}\right)\times\left(2+\frac{4}{7}\right)=6+\left(1+\frac{25}{35}\right)+\frac{28}{35}+\frac{8}{35}=8+\frac{26}{35}}}</math> | |
|- | |- | ||
| | | | ||
| − | :{{#annot:1⁵/₇×1⅘|156|Iwhd}}75) How much are one integer and five sevenths by one integer and four fifths? | + | :{{#annot:1⁵/₇×1⅘|156|Iwhd}}75) If a man asks you: How much are one integer and five sevenths by one integer and four fifths? |
:<math>\scriptstyle\left(1+\frac{5}{7}\right)\times\left(1+\frac{4}{5}\right)</math> | :<math>\scriptstyle\left(1+\frac{5}{7}\right)\times\left(1+\frac{4}{5}\right)</math> | ||
| − | |style="text-align:right;"|עה | + | |style="text-align:right;"|עה <big>אי"א</big> א' וה' שבעיות על א' וד' חמשיות כמה הוא{{#annotend:Iwhd}} |
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{1\sdot1=1}}</math> | + | :Say: 1 time 1 is 1. |
| + | :<math>\scriptstyle{\color{blue}{1\sdot1=1}}</math> | ||
|style="text-align:right;"|אמור א' פעם א' א‫' | |style="text-align:right;"|אמור א' פעם א' א‫' | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{1\sdot\frac{4}{5}=\frac{28}{35}}}</math> | + | :Say: 1 time 4-fifths is 28 parts of 35. |
| − | |style="text-align:right;"|א"כ תאמ' א' פעם ד' חמשיות הם כ"ח חלקי' מל"ה | + | :<math>\scriptstyle{\color{blue}{1\sdot\frac{4}{5}=\frac{28}{35}}}</math> |
| + | |style="text-align:right;"|א"כ תאמ' ‫<ref>95v</ref>א' פעם ד' חמשיות הם כ"ח חלקי' מל"ה | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{1\sdot\frac{5}{7}=\frac{25}{35}}}</math> | + | :Say: 1 time 5-sevenths is 25 parts of 35. |
| + | :<math>\scriptstyle{\color{blue}{1\sdot\frac{5}{7}=\frac{25}{35}}}</math> | ||
|style="text-align:right;"|אח"כ תאמ' א' פעם ה' שבעיות הם כ"ה חלקי' מל"ה | |style="text-align:right;"|אח"כ תאמ' א' פעם ה' שבעיות הם כ"ה חלקי' מל"ה | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\frac{4}{5}\sdot\frac{5}{7}=\frac{20}{35}}}</math> | + | :Then, say: 4-fifths by 5-sevenths is 20 parts of 35. |
| + | :<math>\scriptstyle{\color{blue}{\frac{4}{5}\sdot\frac{5}{7}=\frac{20}{35}}}</math> | ||
|style="text-align:right;"|אחר כן תאמ' ד' חמשיות על ה' שבעיות הם כ' חלקי' מל"ה | |style="text-align:right;"|אחר כן תאמ' ד' חמשיות על ה' שבעיות הם כ' חלקי' מל"ה | ||
|- | |- | ||
| | | | ||
| − | : | + | :Add them up; the result is 3 integers and 3 parts of 35. |
|style="text-align:right;"|צרפם יחד יבא ג' שלמים וג' חלקי' מל"ה וכה"ל | |style="text-align:right;"|צרפם יחד יבא ג' שלמים וג' חלקי' מל"ה וכה"ל | ||
|- | |- | ||
| − | | | + | | colspan="2"| |
| + | :<math>\scriptstyle{\color{blue}{\left(1+\frac{5}{7}\right)\times\left(1+\frac{4}{5}\right)=1+\frac{28}{35}+\frac{25}{35}+\frac{20}{35}=3+\frac{3}{35}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | === <span style=color:green>Multiple Quantities - Coins that Worth an Amount of Money</span> === | ||
| + | |||
| | | | ||
|- | |- | ||
| Line 2,726: | Line 3,235: | ||
:The fourth is equal to four fifths of the third. | :The fourth is equal to four fifths of the third. | ||
:How much is each [of the coins] worth? | :How much is each [of the coins] worth? | ||
| − | + | |style="text-align:right;"|עו <big>הרי</big> שיש לך ד' מטבעות ששוים סך ארבעתם פ' פשוטי‫'<br> | |
| − | |style="text-align:right;"|עו | ||
והראשון שוה החצי והשתות מן השני<br> | והראשון שוה החצי והשתות מן השני<br> | ||
הנשאר מן השני הם ב' שלישיות מן השלשי<br> | הנשאר מן השני הם ב' שלישיות מן השלשי<br> | ||
| Line 2,733: | Line 3,241: | ||
והרבעי הוא ד' חמשים מהשלשי<br> | והרבעי הוא ד' חמשים מהשלשי<br> | ||
ותרצה לידע כמה שוה כל אחד ואחד{{#annotend:7XfF}} | ותרצה לידע כמה שוה כל אחד ואחד{{#annotend:7XfF}} | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle a_1+a_2+a_3+a_4=80\\\scriptstyle a_1=\left(\frac{1}{2}+\frac{1}{6}\right)\sdot a_2\\\scriptstyle a_2-\left[\left(\frac{1}{2}+\frac{1}{6}\right)\sdot a_2\right]=\frac{2}{3}a_3\\\scriptstyle a_3=\left(\frac{1}{3}+\frac{1}{4}+\frac{1}{6}\right)\sdot a_1\\\scriptstyle a_4=\frac{4}{5}a_3\end{cases}</math> | ||
| + | |- | ||
| + | | | ||
| + | :Follow this way: | ||
| + | |style="text-align:right;"|עשה על זה הדרך | ||
| + | |- | ||
| + | | | ||
| + | :Say for the first that is equal to a half and a sixth of the second: | ||
| + | |style="text-align:right;"|אמור הראשון ששוה החצי והשתות מן השני | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Say: a half and a sixth are found in 6: |
| + | |style="text-align:right;"|אמור חצי ושתות אנה ימצא בו‫' | ||
|- | |- | ||
| | | | ||
| − | :::<math>\scriptstyle{\color{blue}{ | + | :The half is 3; the sixth is 1; so they are 4 parts of 6, which are 2-thirds. |
| − | + | |style="text-align:right;"|החצי הוא ג' השתות הוא א' הרי ד' חלקי' מו' שהם ב' שלשיות | |
| − | + | |- | |
| − | + | | colspan="2"| | |
| − | + | :<math>\scriptstyle{\color{blue}{\frac{1}{2}+\frac{1}{6}=\frac{\left(\frac{1}{2}\sdot6\right)+\left(\frac{1}{6}\sdot6\right)}{6}=\frac{3+1}{6}=\frac{4}{6}=\frac{2}{3}}}</math> | |
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{a_1=\left(\frac{1}{2}+\frac{1}{6}\right)\sdot a_2=\frac{2}{3}a_2}}</math> | + | :Therefore, for the one that equals a half and a sixth of the second, it is as if it is said: the first is equal to 2-thirds of the second. |
| + | :<math>\scriptstyle{\color{blue}{a_1=\left(\frac{1}{2}+\frac{1}{6}\right)\sdot a_2=\frac{2}{3}a_2}}</math> | ||
|style="text-align:right;"|א"כ זה ששוה חצי ושתות מן השני הוא כאלו אמר הראשון שוה ב' שלשיות מהשני | |style="text-align:right;"|א"כ זה ששוה חצי ושתות מן השני הוא כאלו אמר הראשון שוה ב' שלשיות מהשני | ||
|- | |- | ||
| | | | ||
| + | :For the third that is equal to a third, a quarter, and a sixth of the first: | ||
|style="text-align:right;"|והשלשי ששוה שליש ורביע ושתות מן הראשון | |style="text-align:right;"|והשלשי ששוה שליש ורביע ושתות מן הראשון | ||
|- | |- | ||
| | | | ||
| − | :::<math>\scriptstyle{\color{blue}{ | + | :Say: a third, a quarter, and a sixth are found in 60: |
| − | + | |style="text-align:right;"|אמור אנה ימצא שליש ורביע ושתות בס‫' | |
| − | + | |- | |
| − | + | | | |
| + | :The third is 20; the quarter is 15; the sixth is 10; so they are 45. | ||
| + | |style="text-align:right;"|השליש הוא כ' הרביע ט"ו והשתות י' הרי למ"ה | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{3}+\frac{1}{4}+\frac{1}{6}=\frac{\left(\frac{1}{3}\sdot60\right)+\left(\frac{1}{4}\sdot60\right)+\left(\frac{1}{6}\sdot60\right)}{60}=\frac{20+15+10}{60}=\frac{45}{60}}}</math> | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{a_3=\frac{45}{60}a_1=\frac{3}{4}a_1}}</math> | + | :Therefore, for the third that is equal to a third, a quarter, and a sixth of the first, which are 45 parts of 60, it is as if it is said: 3-quarters of the first. |
| + | :<math>\scriptstyle{\color{blue}{a_3=\frac{45}{60}a_1=\frac{3}{4}a_1}}</math> | ||
|style="text-align:right;"|א"כ השלשי ששוה שליש ורביע ושתות מן הראשון שהם מ"ה חלקי' מס' הוא כאלו אמר ג' רבעיות מן הראשון | |style="text-align:right;"|א"כ השלשי ששוה שליש ורביע ושתות מן הראשון שהם מ"ה חלקי' מס' הוא כאלו אמר ג' רבעיות מן הראשון | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{a_4=\frac{4}{5}a_3}}</math> | + | :For the fourth that is equal to 4-fifths of the third - it is clear. |
| + | :<math>\scriptstyle{\color{blue}{a_4=\frac{4}{5}a_3}}</math> | ||
|style="text-align:right;"|והרבעי ששוה ד' חמשיות מהשלשי הוא מבואר | |style="text-align:right;"|והרבעי ששוה ד' חמשיות מהשלשי הוא מבואר | ||
|- | |- | ||
| | | | ||
| − | :: | + | :Therefore, this is the order: the first equals 2-thirds of the second; the third equals 3-quarters of the first; and the fourth equals 4-fifths of the third. |
|style="text-align:right;"|א"כ הסדר כך הוא הראשון ששוה ב' שלשיות מהשני<br> | |style="text-align:right;"|א"כ הסדר כך הוא הראשון ששוה ב' שלשיות מהשני<br> | ||
והשלשי שוה ג' רבעיות מן הראשון<br> | והשלשי שוה ג' רבעיות מן הראשון<br> | ||
והרבעי שוה ד' חמשים מהשלשי | והרבעי שוה ד' חמשים מהשלשי | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | :<math>\scriptstyle{\color{blue}{a_1=\frac{2}{3}a_2\quad a_3=\frac{3}{4} a_1\quad a_4=\frac{4}{5}a_3}}</math> | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''False Position:'''</span> First say: the fractions mentioned in this calculation, which are a half, a third, a quarter, a fifth, and a sixth, are all found in 60. | |
| + | :<math>\scriptstyle{\color{blue}{b_2=60}}</math> | ||
|style="text-align:right;"|אמור תחלה השברים הנז' בזה החשבון הם חצי ושליש ורבע וחומש ושתות וימצאו כלם בס‫' | |style="text-align:right;"|אמור תחלה השברים הנז' בזה החשבון הם חצי ושליש ורבע וחומש ושתות וימצאו כלם בס‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | :Hence, the second is 60. |
| − | |style="text-align:right;"|א"כ השני הוא ס | + | |style="text-align:right;"|א"כ השני הוא ס‫' |
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :The first that is equal to 2-thirds of the second, which is 60, is 40, because 40 is 2-thirds of 60. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והראשון שהוא שוה ב' שלשיות מן השני שהוא ס' הוא מ' כי מ' הוא ב' שלשיות מס‫' |
| − | |||
| − | |||
| − | כי | ||
|- | |- | ||
| | | | ||
| − | ::<span style=color: | + | :Then, the third that is equal to 3-quarters of the first, which is 40, is 30, because 30 is 3-quarters of 40. |
| + | |style="text-align:right;"|והשלשי ששוה ג' רבעיות מהראשון שהוא מ' א"כ הוא ל' כי ל' הם ג' רבעיות ממ‫' | ||
| + | |- | ||
| + | | | ||
| + | :The fourth that is equal to 4-fifths of the third, which is 30, is 24, because 24 is 4-fifths of 30. | ||
| + | |style="text-align:right;"|והרבעי ששוה ד' חמשיות מהשלשי שהוא ל' הוא כ"ד כי כ"ד הוא ד' חמשיות מל‫' | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | :<math>\scriptstyle{\color{blue}{\begin{cases}\scriptstyle b_2=60\\\scriptstyle b_1=\frac{2}{3}b_2=\frac{2}{3}\sdot60=40\\\scriptstyle b_3=\frac{3}{4}b_1=\frac{3}{4}\sdot40=30\\\scriptstyle b_4=\frac{4}{5}b_3=\frac{4}{5}\sdot30=24\end{cases}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :For what is left of the second that is 2-thirds of third, follow this way: | ||
| + | :<math>\scriptstyle{\color{blue}{b_2-b_1=60-40=20=\frac{2}{3}\sdot30=\frac{2}{3}b_3}}</math> | ||
| + | |style="text-align:right;"|והנשאר מהשני שהוא ב' שלשיות מהשלשי עשה על זה הדרך | ||
| + | |- | ||
| + | | | ||
| + | :Say: how much is left of the second, which is 60, after you subtract the first from it, which is 40? 20 remain. | ||
| + | |style="text-align:right;"|אמור כמה נשאר מן השני שהוא ס' אחר שהוצאת ממנו הראשון שהוא מ' נשארו כ‫' | ||
| + | |- | ||
| + | | | ||
| + | :So, 20, which is what is left of the second, is 2-thirds of the third, which is 30, because 20 is 2-thirds of 30. | ||
| + | |style="text-align:right;"|א"כ כ' שהוא הנשאר מהשני הם ב' שלשיות מן השלשי שהוא ל' כי כ' הם ב' שלשיות מל‫' | ||
| + | |- | ||
| + | | | ||
| + | :<span style=color:green>'''Denominator:'''</span> So, the first is 40; the second is 60; the third - 30; and the fourth - 24. Sum them together; the result is 154 and this is the denominator. | ||
| + | :<math>\scriptstyle{\color{blue}{b_1+b_2+b_3+b_4=40+60+30+24=154}}</math> | ||
|style="text-align:right;"|הרי שהראשון הוא מ' והשני הוא ס' והשלשי ל' והרבעי כ"ד צרף אותם יחד יעלו קנ"ד והוא המחלק | |style="text-align:right;"|הרי שהראשון הוא מ' והשני הוא ס' והשלשי ל' והרבעי כ"ד צרף אותם יחד יעלו קנ"ד והוא המחלק | ||
|- | |- | ||
| | | | ||
| − | : | + | :<span style=color:green>'''Rule of Three:'''</span> If you wish to know how much the first, which is 40, is worth, say: 40 times 80, because they are worth together 80; it is 3200. Divide it by 154; the result is 20 and 120 parts of 154. |
| + | :<math>\scriptstyle{\color{blue}{a_1=\frac{40\sdot80}{154}=\frac{3200}{154}=20+\frac{120}{154}}}</math> | ||
|style="text-align:right;"|ואם תרצה לידע כמה שוה הראשון שהוא מ‫'<br> | |style="text-align:right;"|ואם תרצה לידע כמה שוה הראשון שהוא מ‫'<br> | ||
אמור מ' פעמ' פ' בעבור שכלם שוים פ' יהיו ג' אלפי' ומאתיים<br> | אמור מ' פעמ' פ' בעבור שכלם שוים פ' יהיו ג' אלפי' ומאתיים<br> | ||
| Line 2,800: | Line 3,353: | ||
|- | |- | ||
| | | | ||
| − | : | + | :Do the same for the others and you will get the same answer. |
|style="text-align:right;"|ובזה הדרך תעשה מן האחרים ויצא לך הענין השוה וכה"ל | |style="text-align:right;"|ובזה הדרך תעשה מן האחרים ויצא לך הענין השוה וכה"ל | ||
|- | |- | ||
| − | | | + | | |
| + | |||
| + | === <span style=color:green>Find a Number Problems - Sums</span> === | ||
| + | |||
| | | | ||
|- | |- | ||
| | | | ||
| − | :{{#annot:1-8|669|zy8k}}77) 1, 2, 3, 4, 5, 6, 7, 8 | + | :{{#annot:1-8|669|zy8k}}77) 1, 2, 3, 4, 5, 6, 7, 8. |
:<math>\scriptstyle1+2+3+4+5+6+7+8=\sum_{i=1}^{8} i</math> | :<math>\scriptstyle1+2+3+4+5+6+7+8=\sum_{i=1}^{8} i</math> | ||
| − | |style="text-align:right;"|עז | + | |style="text-align:right;"|עז <big>א</big> ב' ג' ד' ה' ו' ז' ח‫'{{#annotend:zy8k}} |
|- | |- | ||
| | | | ||
| − | :[ | + | :[The number of items] can be divided in half [= last term is even <math>\scriptstyle{\color{OliveGreen}{n=2m}}</math>]: |
|style="text-align:right;"|שהם נחלקי' לחצי | |style="text-align:right;"|שהם נחלקי' לחצי | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle\sum_{i=1}^n i=\frac{1}{2}n\sdot\left(n+1\right)</math> | + | :Add 1 to the [last number] and multiply it by its half. |
| + | :<math>\scriptstyle{\color{OliveGreen}{\sum_{i=1}^n i=\frac{1}{2}n\sdot\left(n+1\right)}}</math> | ||
|style="text-align:right;"|תוסיף א' על הסך ותרבה אותו על החצי | |style="text-align:right;"|תוסיף א' על הסך ותרבה אותו על החצי | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\sum_{i=1}^8 i=\left(\frac{1}{2}\sdot8\right)\sdot\left(8+1\right)=4\sdot9=36}}</math> | + | :Say: 4 times 9 is 36; so their sum is 36. |
| + | :<math>\scriptstyle{\color{blue}{\sum_{i=1}^8 i=\left(\frac{1}{2}\sdot8\right)\sdot\left(8+1\right)=4\sdot9=36}}</math> | ||
|style="text-align:right;"|ואמור ד' פעמ' ט' ל"ו וכן יהיה מספרם ל"ו וכה"ל | |style="text-align:right;"|ואמור ד' פעמ' ט' ל"ו וכן יהיה מספרם ל"ו וכה"ל | ||
|- | |- | ||
| | | | ||
| − | :{{#annot:1-7|669|bsMG}}78) 1, 2, 3, 4, 5, 6, 7 | + | :{{#annot:1-7|669|bsMG}}78) 1, 2, 3, 4, 5, 6, 7. |
:<math>\scriptstyle1+2+3+4+5+6+7=\sum_{i=1}^{7} i</math> | :<math>\scriptstyle1+2+3+4+5+6+7=\sum_{i=1}^{7} i</math> | ||
| − | |style="text-align:right;"|עח | + | |style="text-align:right;"|עח <big>א</big> ב' ג' ד' ה' ו' ז‫'{{#annotend:bsMG}} |
|- | |- | ||
| | | | ||
| − | :[ | + | :[The number of items] cannot be divided in half [= last term is odd <math>\scriptstyle{\color{OliveGreen}{n=2m+1}}</math>]: |
|style="text-align:right;"|שאינם נחלקים לחצי | |style="text-align:right;"|שאינם נחלקים לחצי | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle\sum_{i=1}^n i=\left[\frac{1}{2}\sdot\left(n+1\right)\right]\sdot n</math> | + | :Take the greater half and multiply it by the last number. |
| + | :<math>\scriptstyle{\color{OliveGreen}{\sum_{i=1}^n i=\left[\frac{1}{2}\sdot\left(n+1\right)\right]\sdot n}}</math> | ||
|style="text-align:right;"|תפוס הרוב ותרבה אותו על הסך | |style="text-align:right;"|תפוס הרוב ותרבה אותו על הסך | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\sum_{i=1}^7 i=\left[\frac{1}{2}\sdot\left(7+1\right)\right]\sdot7=4\sdot7=28}}</math> | + | :Say: 4 times 7 is 28. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{\sum_{i=1}^7 i=\left[\frac{1}{2}\sdot\left(7+1\right)\right]\sdot7=4\sdot7=28}}</math> |
| + | |style="text-align:right;"|ואמור ד' פעמ' ז' כ"ח וכה"ל | ||
|- | |- | ||
| | | | ||
| − | :{{#annot:1-9|670|djRx}}79) 1, 3, 5, 7, 9 - that are | + | :{{#annot:1-9|670|djRx}}79) 1, 3, 5, 7, 9 - that are odds. |
:<math>\scriptstyle1+3+5+7+9=\sum_{i=1}^{5} \left(2i-1\right)</math> | :<math>\scriptstyle1+3+5+7+9=\sum_{i=1}^{5} \left(2i-1\right)</math> | ||
| − | |style="text-align:right;"|עט | + | |style="text-align:right;"|עט <big>א</big> ג' ה' ז' ט' שהם פרדים{{#annotend:djRx}} |
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle\sum_{i=1}^n \left(2i-1\right)=n^2</math> | + | :Multiply the greater half by itself. |
| + | :<math>\scriptstyle{\color{OliveGreen}{\sum_{i=1}^n \left(2i-1\right)=n^2}}</math> | ||
|style="text-align:right;"|תרבה הרוב בעצמו | |style="text-align:right;"|תרבה הרוב בעצמו | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\sum_{i=1}^5 \left(2i-1\right)=5^2=25}}</math> | + | :Say: the greater half of 9 is 5. Say 5 times 5 is 25 and this is their sum. |
| + | :<math>\scriptstyle{\color{blue}{\sum_{i=1}^5 \left(2i-1\right)=5^2=25}}</math> | ||
|style="text-align:right;"|ואמור הרוב מט' הוא ה' ואמ' ה' פעמ' ה' כ"ה וכן יבאו וכה"ל | |style="text-align:right;"|ואמור הרוב מט' הוא ה' ואמ' ה' פעמ' ה' כ"ה וכן יבאו וכה"ל | ||
|- | |- | ||
| | | | ||
| − | :{{#annot:2-8|671|zzdV}}80) 2, 4, 6, 8 - that are | + | :{{#annot:2-8|671|zzdV}}80) 2, 4, 6, 8 - that are evens. |
:<math>\scriptstyle2+4+6+8=\sum_{i=1}^{4} 2i</math> | :<math>\scriptstyle2+4+6+8=\sum_{i=1}^{4} 2i</math> | ||
| − | |style="text-align:right;"|פ | + | |style="text-align:right;"|פ <big>ב</big> ד' ו' ח' שהם כלם זוגות{{#annotend:zzdV}} |
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle\sum_{i=1}^n 2i=n\sdot\left(n+1\right)</math> | + | :Multiply their half by the number that follows it. |
| + | :<math>\scriptstyle{\color{OliveGreen}{\sum_{i=1}^n 2i=n\sdot\left(n+1\right)}}</math> | ||
|style="text-align:right;"|תרבה חציים על הבא לאחריו | |style="text-align:right;"|תרבה חציים על הבא לאחריו | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\sum_{i=1}^4 2i=4\sdot\left(4+1\right)=4\sdot5=20}}</math> | + | :Say: half 8 is 4. Say: 4 times 5 is 20 and this is their sum. |
| − | |style="text-align:right;"|ואמור החצי מח' ד' ואמור ד' פעמ' ה' הם כ' וכן יבא וכה"ל | + | :<math>\scriptstyle{\color{blue}{\sum_{i=1}^4 2i=4\sdot\left(4+1\right)=4\sdot5=20}}</math> |
| + | |style="text-align:right;"|ואמור החצי מח' ד' ‫<ref>96r</ref>ואמור ד' פעמ' ה' הם כ' וכן יבא וכה"ל | ||
|- | |- | ||
| − | |||
| | | | ||
| − | |- | + | |
| + | === <span style=color:green>Find a Number Problem - Multiplication of integers and fractions</span> === | ||
| + | |||
| + | | | ||
| + | |- | ||
| | | | ||
| − | :{{#annot:1¼×1⅕|156|uW3p}}81) How much are one integer and a quarter by one integer and a fifth? | + | :{{#annot:1¼×1⅕|156|uW3p}}81) If a man asks you: How much are one integer and a quarter by one integer and a fifth? |
:<math>\scriptstyle\left(1+\frac{1}{4}\right)\times\left(1+\frac{1}{5}\right)</math> | :<math>\scriptstyle\left(1+\frac{1}{4}\right)\times\left(1+\frac{1}{5}\right)</math> | ||
| − | |style="text-align:right;"|פא | + | |style="text-align:right;"|פא <big>אם</big> ישאלך אדם א' ורביע על א' וחמישית כמה הוא{{#annotend:uW3p}} |
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{1\sdot1=1}}</math> | + | :First, say: 1 time 1 is 1. |
| + | :<math>\scriptstyle{\color{blue}{1\sdot1=1}}</math> | ||
|style="text-align:right;"|אמור תחלה א' פעם א' א‫' | |style="text-align:right;"|אמור תחלה א' פעם א' א‫' | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\left(1\sdot\frac{1}{4}\right)+\left(1\sdot\frac{1}{5}\right)=\frac{1}{4}+\frac{1}{5}=\frac{9}{20}}}</math> | + | :Then, say: one time a quarter and one times a fifth are 9-twentieths. |
| + | :<math>\scriptstyle{\color{blue}{\left(1\sdot\frac{1}{4}\right)+\left(1\sdot\frac{1}{5}\right)=\frac{1}{4}+\frac{1}{5}=\frac{9}{20}}}</math> | ||
|style="text-align:right;"|אח"כ תאמ' פעם אחת רביע ופעם אחת חמישית הם ט' בנטיני‫' | |style="text-align:right;"|אח"כ תאמ' פעם אחת רביע ופעם אחת חמישית הם ט' בנטיני‫' | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\frac{1}{4}\sdot\frac{1}{5}=\frac{1}{20}}}</math> | + | :Say: a quarter by a fifth is 1 part of 20. |
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{4}\sdot\frac{1}{5}=\frac{1}{20}}}</math> | ||
|style="text-align:right;"|אחר כך תאמ' א' רביע על א' חמישית הוא א' חלק מכ‫' | |style="text-align:right;"|אחר כך תאמ' א' רביע על א' חמישית הוא א' חלק מכ‫' | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\left(1+\frac{1}{4}\right)\times\left(1+\frac{1}{5}\right)=1+\frac{9}{20}+\frac{1}{20}=1+\frac{10}{20}=1+\frac{1}{2}}}</math> | + | :We find that the total is 1 and 10-twentieths, i.e. 1 and a half. |
| − | |style="text-align:right;"|נמצא שיבא הכל א' וי' בנטיני' דהיינו א' וחצי | + | :<math>\scriptstyle{\color{blue}{\left(1+\frac{1}{4}\right)\times\left(1+\frac{1}{5}\right)=1+\frac{9}{20}+\frac{1}{20}=1+\frac{10}{20}=1+\frac{1}{2}}}</math> |
| + | |style="text-align:right;"|נמצא שיבא <sup>הכל</sup> א' וי' בנטיני' דהיינו א' וחצי | ||
|- | |- | ||
| − | | | + | | |
| + | |||
| + | === <span style=color:green>Interest and Discount Problems</span> === | ||
| + | |||
| | | | ||
|- | |- | ||
| − | | | + | | |
| + | ==== <span style=color:green>Find the Earned Interest</span> ==== | ||
| + | |||
| | | | ||
|- | |- | ||
| | | | ||
| − | :{{#annot:for some liṭra a year|638|YSZs}}82) | + | :{{#annot:for some liṭra a year|638|YSZs}}82) If a man asks you: I lent some money at so and so pešuṭim for a liṭra a month, how much is the profit of 100 liṭra for a year? |
| − | + | :<math>\scriptstyle\frac{a}{1}=\frac{20\sdot X}{100}</math> | |
| − | :<math>\scriptstyle\frac{a}{1 | + | |style="text-align:right;"|פב <big>אם</big> ישאלך אדם הלותי מעות לחשבון כך וכך פשו' החדש הליט‫'<br> |
| − | |style="text-align:right;"|פב | ||
כמה יעלה ריוח בק' ליט' א' שנה{{#annotend:YSZs}} | כמה יעלה ריוח בק' ליט' א' שנה{{#annotend:YSZs}} | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle X=a\sdot5</math> | + | :Multiply the money that the liṭra yields per month by 5. |
| + | :<math>\scriptstyle{\color{OliveGreen}{X=a\sdot5}}</math> | ||
|style="text-align:right;"|תרבה ה' פעמ' כנגד המעות שירויח הליט' החדש | |style="text-align:right;"|תרבה ה' פעמ' כנגד המעות שירויח הליט' החדש | ||
|- | |- | ||
| | | | ||
| − | + | *{{#annot:for some liṭra a year|638|YKRT}}Example: I lent 100 liṭra for one [year] at 7 pešuṭim for one liṭra a month. | |
| − | + | :<math>\scriptstyle\frac{7}{1}=\frac{20\sdot X}{100}</math> | |
|style="text-align:right;"|המשל בזה הרי שהלוית ק' ליט' א' חדש לחשבון ז"פ החדש הליט‫'{{#annotend:YKRT}} | |style="text-align:right;"|המשל בזה הרי שהלוית ק' ליט' א' חדש לחשבון ז"פ החדש הליט‫'{{#annotend:YKRT}} | ||
|- | |- | ||
| | | | ||
| − | :::<math>\scriptstyle{\color{blue}{x=5\sdot7=35}}</math> | + | :Say: 5 times 7 is 35. |
| − | |style="text-align:right;"|תאמ' ה' פעמ' ז' ל"ה | + | :<math>\scriptstyle{\color{blue}{x=5\sdot7=35}}</math> |
| + | |style="text-align:right;"|תאמ' ה' פעמ' ז' ל"ה | ||
|- | |- | ||
| − | |||
| | | | ||
| + | :We find that 100 liṭra yield 35 liṭra a year. | ||
| + | |style="text-align:right;"|נמצא שיבא הק' ליט' השנה ל"ה ליט' וכה"ל | ||
|- | |- | ||
| | | | ||
| − | + | ||
| − | + | ==== <span style=color:green>Compound Interest</span> ==== | |
| + | |||
| + | | | ||
|- | |- | ||
| | | | ||
| − | : | + | :{{#annot:WP|640|hntq}}83) The one who wants to combine several loans made at different times and convert them to one time: |
| + | |style="text-align:right;"|פג) <big>מי</big> שרוצה לקבץ חשבונות הלוואות רבים שנעשו בזמנים שונים להביאם בזמן אחד{{#annotend:hntq}} | ||
| + | |- | ||
| + | | | ||
| + | :He should sum up all the monthly interests and divide [the sum] by the amount of money, or peraḥim, or any currency [specified] for those loans. | ||
|style="text-align:right;"|יקבץ חשבונות החדשים כלם ויחלקם כחשבון המעות או הפרחי' או כמטבע ההלוואות ההם | |style="text-align:right;"|יקבץ חשבונות החדשים כלם ויחלקם כחשבון המעות או הפרחי' או כמטבע ההלוואות ההם | ||
|- | |- | ||
| | | | ||
| − | + | *{{#annot:WP|640|RZRA}}Example: I lent 100 peraḥim of gold for a month, 100 peraḥim for two months, 100 peraḥim for three months, 100 peraḥim for four months, and 100 peraḥim for five months | |
|style="text-align:right;"|המשל בזה הרי שהלוית ק' פרחי' זהב א' חדש וק' פרחי' ב' חדשים וק' פרחי' ג' חדשי' וק' פרחי' ד' חדשי' וק' פרחי' ה' חדשי‫'{{#annotend:RZRA}} | |style="text-align:right;"|המשל בזה הרי שהלוית ק' פרחי' זהב א' חדש וק' פרחי' ב' חדשים וק' פרחי' ג' חדשי' וק' פרחי' ד' חדשי' וק' פרחי' ה' חדשי‫'{{#annotend:RZRA}} | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\frac{1+2+3+4+5}{ | + | :Do as follows: one, two, three, four, five - which is [the number of] months - is 15. |
| − | + | |style="text-align:right;"|עשה כן אחד ושנים ושלשה וארבעה וחמשה שהם החדשי' הרי ט"ו | |
| − | |style="text-align:right;"| | + | |- |
| − | חלקם בסכום המעות שהם ה' יגיע לכל חלק מהם ג‫' | + | | |
| − | הרי שעמדו כלם ג' חדשי‫' | + | :Divide it by the amount of money, which is 5; the quotient is 3. |
| + | :<math>\scriptstyle{\color{blue}{\frac{1+2+3+4+5}{\frac{100+100+100+100+100}{100}}=\frac{15}{5}=3}}</math> | ||
| + | |style="text-align:right;"|חלקם בסכום המעות שהם ה' יגיע לכל חלק מהם ג‫' | ||
| + | |- | ||
| + | | | ||
| + | :So, all are set for 3 months, | ||
| + | |style="text-align:right;"|הרי שעמדו כלם ג' חדשי‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|וכן הדומה לכל מטבע ולכל | + | :The same for every currency and every number. |
| + | |style="text-align:right;"|וכן הדומה לכל מטבע ולכל מנין וכה"ל | ||
|- | |- | ||
| − | | | + | | |
| + | |||
| + | ==== <span style=color:green>Find the Earned Interest</span> ==== | ||
| + | |||
| | | | ||
|- | |- | ||
| | | | ||
| − | :{{#annot:for some liṭra a month|638|HIJ5}}84) | + | :{{#annot:for some liṭra a month|638|HIJ5}}84) The one who wants to know for one kikkar at so and so [a year], how much will be [the interest] a month? |
| − | + | :<math>\scriptstyle\frac{20\sdot a}{100}=\frac{X}{b}</math> | |
| − | :<math>\scriptstyle\frac{ | + | |style="text-align:right;"|פד <big>הרוצה</big> לידע לחשבון כך וכך הככר כמה יבואו קצתם לחדש{{#annotend:HIJ5}} |
| − | |style="text-align:right;"|פד | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> multiply the interest of the kikkar by the amount of money you want, then divide by five and the resulting quotient is [the interest] per month for the amount you want. | |
| + | :<math>\scriptstyle{\color{OliveGreen}{X=\frac{b\sdot a}{5}}}</math> | ||
|style="text-align:right;"|תרבה חשבון הככר כסכום המעות שתרצה וחלקם בחמשה והחלק שיצא יגיע לכל חדש כסכום ההוא | |style="text-align:right;"|תרבה חשבון הככר כסכום המעות שתרצה וחלקם בחמשה והחלק שיצא יגיע לכל חדש כסכום ההוא | ||
|- | |- | ||
| | | | ||
| − | + | *{{#annot:for some liṭra a month|638|ErCS}}Example: At 7 liṭra for one kikkar a year: how much will 30 liṭra yield a month or two months or more? | |
| − | : | + | :<math>\scriptstyle\frac{20\sdot7}{100}=\frac{X}{30}</math> |
| − | + | |style="text-align:right;"|המשל בזה לחשבון ז' ליט' הככר לשנה כמה יבא ל' ליט' חדש אחד או ב' חדשי' או יותר{{#annotend:ErCS}} | |
| − | |style="text-align:right;"|המשל בזה לחשבון ז' ליט' הככר לשנה כמה יבא | + | |- |
| + | | | ||
| + | :Multiply 30 by 7; it is 210. | ||
| + | |style="text-align:right;"|תרבה ל' בז' והם ר"י | ||
| + | |- | ||
| + | | | ||
| + | :Divide by five; each part is 42. | ||
| + | :<math>\scriptstyle{\color{blue}{x=\frac{30\sdot7}{5}=\frac{210}{5}=42}}</math> | ||
| + | |style="text-align:right;"|חלקם בחמשה יגיע לכל חלק מהם מ"ב | ||
|- | |- | ||
| | | | ||
| − | : | + | :So, [the interest is] 42 pešuṭim a month. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|הרי שבמב"פ יבא לכל לחדש אחד |
| − | |||
| − | הרי שבמב"פ יבא לכל לחדש אחד | ||
|- | |- | ||
| | | | ||
| + | :Then, multiply by [the number of] months you want and you will get the answer. | ||
|style="text-align:right;"|אחרי כן תרבה החדשים שתרצה ויצא לך הענין וכה"ל | |style="text-align:right;"|אחרי כן תרבה החדשים שתרצה ויצא לך הענין וכה"ל | ||
|- | |- | ||
| − | | | + | | |
| + | |||
| + | === <span style=color:green>Multiple Quantities Problem - Five Weights</span> === | ||
| + | |||
| | | | ||
|- | |- | ||
| | | | ||
| − | :{{#annot:five weights| | + | :{{#annot:five weights|652|Z0uf}}85) The butcher sells meat but he has only five weights summed up together to 121 liṭra. |
:How much should each of the weights weigh, so that the butcher will be able to weigh the meat with these weights be it light or heavy? | :How much should each of the weights weigh, so that the butcher will be able to weigh the meat with these weights be it light or heavy? | ||
:<math>\scriptstyle\begin{cases}\scriptstyle\sum_{i=1}^5 a_i=121\\\scriptstyle a_i=3^{i-1}\\\scriptstyle a_i:a_{i+1}=a_{i+1}:a_{i+2}\end{cases}</math> | :<math>\scriptstyle\begin{cases}\scriptstyle\sum_{i=1}^5 a_i=121\\\scriptstyle a_i=3^{i-1}\\\scriptstyle a_i:a_{i+1}=a_{i+1}:a_{i+2}\end{cases}</math> | ||
| − | |style="text-align:right;"|פה | + | |style="text-align:right;"|פה <big>אם</big> הקצב מוכר בשר ואין לו רק ה' משקלות ששוקלות בין כולם קכ"א ליט‫'<br> |
כמה ישקול כל משקל לעצמו שיוכל הקצב לשקול הבשר באלו המשקלות למי רב ולמי מעט{{#annotend:Z0uf}} | כמה ישקול כל משקל לעצמו שיוכל הקצב לשקול הבשר באלו המשקלות למי רב ולמי מעט{{#annotend:Z0uf}} | ||
|- | |- | ||
| | | | ||
| − | : | + | :So, if someone wants to buy 121 liṭra of meat, he puts all the weights against the meat. |
|style="text-align:right;"|שמי שירצה לקנות קכ"א ליט' בשר ישים כל המשקלות כנגד הבשר | |style="text-align:right;"|שמי שירצה לקנות קכ"א ליט' בשר ישים כל המשקלות כנגד הבשר | ||
|- | |- | ||
| | | | ||
| − | : | + | :If he wants to buy 50 liṭra of meat, or more or less, he puts as many weights in the scales against the meat, so that they will weigh as much as the meat he wants to buy. |
|style="text-align:right;"|ואם ירצה לקנות נ' ליט' בשר או יותר או פחות ישים כל כך משקלות בצד המאזנים שכנגד הבשר שישקלו כמו הבשר שהוא רוצה לקנות | |style="text-align:right;"|ואם ירצה לקנות נ' ליט' בשר או יותר או פחות ישים כל כך משקלות בצד המאזנים שכנגד הבשר שישקלו כמו הבשר שהוא רוצה לקנות | ||
| + | |- | ||
| + | | | ||
| + | :If there is no balance, he changes the weights. | ||
| + | |style="text-align:right;"|ואם לא יבא בכיוון יחליף המשקלות | ||
|- | |- | ||
| | | | ||
| − | : | + | :Sometimes he must put one, or two, or three weights with the meat against one weight to fulfill the buyer's wish. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ולפעמים צריך שישים אחד ושנים ושלשה משקלות עם הבשר כנגד משקל אחד להפיק רצון הקונה |
|- | |- | ||
| | | | ||
| + | :Now, I ask you: How much does each weight weigh by itself? | ||
|style="text-align:right;"|ועתה אשאלך כמה ישקול כל משקל לעצמו | |style="text-align:right;"|ועתה אשאלך כמה ישקול כל משקל לעצמו | ||
|- | |- | ||
| | | | ||
| − | :: | + | :One weighs 1 liṭra. |
| + | :<math>\scriptstyle{\color{blue}{a_1=1}}</math> | ||
|style="text-align:right;"|האחד ישקול א' ליט‫' | |style="text-align:right;"|האחד ישקול א' ליט‫' | ||
|- | |- | ||
| | | | ||
| − | :: | + | :The second weighs 3 liṭra. |
| + | :<math>\scriptstyle{\color{blue}{a_2=3}}</math> | ||
|style="text-align:right;"|והשני ישקול ג' ליט‫' | |style="text-align:right;"|והשני ישקול ג' ליט‫' | ||
|- | |- | ||
| | | | ||
| − | :: | + | :The third - 9 liṭra. |
| + | :<math>\scriptstyle{\color{blue}{a_3=9}}</math> | ||
|style="text-align:right;"|והשלישי ט' ליט‫' | |style="text-align:right;"|והשלישי ט' ליט‫' | ||
|- | |- | ||
| | | | ||
| − | :: | + | :The fourth - 27 liṭra. |
| + | :<math>\scriptstyle{\color{blue}{a_4=27}}</math> | ||
|style="text-align:right;"|והרבעי כ"ז ליט‫' | |style="text-align:right;"|והרבעי כ"ז ליט‫' | ||
|- | |- | ||
| | | | ||
| − | :: | + | :The fifth - 81 liṭra. |
| + | :<math>\scriptstyle{\color{blue}{a_5=81}}</math> | ||
|style="text-align:right;"|והחמשי פ"א ליט‫' | |style="text-align:right;"|והחמשי פ"א ליט‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | :Add them together; they are 121 liṭra, no less and no more. |
| + | :<math>\scriptstyle{\color{blue}{1+3+9+27+81=121}}</math> | ||
|style="text-align:right;"|צרפם יחד יהיו קכ"א ליט' לא פחות ולא יותר | |style="text-align:right;"|צרפם יחד יהיו קכ"א ליט' לא פחות ולא יותר | ||
|- | |- | ||
| | | | ||
| + | :With these weights the butcher can sell as he wishes, to some a lot and to some a little. | ||
|style="text-align:right;"|ובאלו המשקלות יוכל הקצב למכור כרצונו למי רב ולמי מעט וכה"ל | |style="text-align:right;"|ובאלו המשקלות יוכל הקצב למכור כרצונו למי רב ולמי מעט וכה"ל | ||
|- | |- | ||
| − | | | + | | |
| + | |||
| + | === <span style=color:green>Guessing - coins</span> === | ||
| + | |||
| | | | ||
|- | |- | ||
| | | | ||
| − | :{{#annot:coins|667|yG7H}}86) Three different coins [gold, silver, and copper, given] to three different people: give the first one pašuṭ, the second two [pešuṭim], and the third four [pešuṭim]. | + | :{{#annot:three coins|667|yG7H}}86) Three different coins [gold, silver, and copper, given] to three different people: give the first one pašuṭ, the second two [pešuṭim], and the third four [pešuṭim]. |
:The one who has the largest coin should multiply his share [= the number of the pešuṭim he has] by 4, the second by 3, and the one who has the smallest coin should double his share once. | :The one who has the largest coin should multiply his share [= the number of the pešuṭim he has] by 4, the second by 3, and the one who has the smallest coin should double his share once. | ||
:Then they should sum up [the products] and cast out the sevens from the result | :Then they should sum up [the products] and cast out the sevens from the result | ||
| − | |style="text-align:right;"|פו | + | |style="text-align:right;"|פו <big>חשבון</big> הג' מטבעות שונות לג' אנשים שונים להגידם לראשון תן א"פ ולשני ב' ולשלשי ד‫'<br> |
| − | ובעל המטבע הגדול יכפול חלקו ד' פעמ' והשני ג' פעמים ובעל המטבע הקטן יכפול חלקו פעם אחד<br> | + | ובעל המטבע הגדול יכפול חלקו ד' פעמ' והשני ג' פעמים ‫<ref>96v</ref>ובעל המטבע הקטן יכפול חלקו פעם אחד<br> |
ויקבצום וישלכום ז"ז{{#annotend:yG7H}} | ויקבצום וישלכום ז"ז{{#annotend:yG7H}} | ||
|- | |- | ||
| | | | ||
| − | : | + | :<math>\scriptstyle{\color{OliveGreen}{\left[\left(2\sdot1\right)+\left(4\sdot2\right)+\left(3\sdot4\right)\right]\bmod7=}}</math> 1 → copper, gold, silver. |
|style="text-align:right;"|וסימן א' כסף זהב נחשת | |style="text-align:right;"|וסימן א' כסף זהב נחשת | ||
|- | |- | ||
| | | | ||
| − | : | + | :<math>\scriptstyle{\color{OliveGreen}{\left[\left(3\sdot1\right)+\left(2\sdot2\right)+\left(4\sdot4\right)\right]\bmod7=}}</math> 2 → silver, copper, gold. |
|style="text-align:right;"|ב' זהב נחשת כסף | |style="text-align:right;"|ב' זהב נחשת כסף | ||
|- | |- | ||
| | | | ||
| − | : | + | :<math>\scriptstyle{\color{OliveGreen}{\left[\left(2\sdot1\right)+\left(3\sdot2\right)+\left(4\sdot4\right)\right]\bmod7=}}</math> 3 → copper, silver, gold. |
|style="text-align:right;"|ג' זהב כסף נחשת | |style="text-align:right;"|ג' זהב כסף נחשת | ||
|- | |- | ||
| | | | ||
| − | : | + | :<math>\scriptstyle{\color{OliveGreen}{\left[\left(4\sdot1\right)+\left(3\sdot2\right)+\left(2\sdot4\right)\right]\bmod7=}}</math> 4 → gold, silver, copper. |
|style="text-align:right;"|ד' נחשת כסף זהב | |style="text-align:right;"|ד' נחשת כסף זהב | ||
|- | |- | ||
| | | | ||
| − | : | + | :<math>\scriptstyle{\color{OliveGreen}{\left[\left(3\sdot1\right)+\left(4\sdot2\right)+\left(2\sdot4\right)\right]\bmod7=}}</math> 5 → silver, gold, copper. |
|style="text-align:right;"|ה' נחשת זהב כסף | |style="text-align:right;"|ה' נחשת זהב כסף | ||
|- | |- | ||
| | | | ||
| − | : | + | :<math>\scriptstyle{\color{OliveGreen}{\left[\left(4\sdot1\right)+\left(2\sdot2\right)+\left(3\sdot4\right)\right]\bmod7=}}</math> 6 → gold, copper, silver. |
|style="text-align:right;"|ו' כסף נחשת זהב וכה"ל | |style="text-align:right;"|ו' כסף נחשת זהב וכה"ל | ||
|- | |- | ||
| − | | | + | | |
| + | |||
| + | === <span style=color:green>Payment Problem - three workers, three different daily wages, the same actual payment</span> === | ||
| + | |||
| | | | ||
|- | |- | ||
| Line 3,066: | Line 3,688: | ||
:How much is the share of each of them? | :How much is the share of each of them? | ||
:<math>\scriptstyle\begin{cases}\scriptstyle\frac{5}{20}X=\frac{4}{20}Y=\frac{3}{20}Z\\\scriptstyle X+Y+Z=20\end{cases}</math> | :<math>\scriptstyle\begin{cases}\scriptstyle\frac{5}{20}X=\frac{4}{20}Y=\frac{3}{20}Z\\\scriptstyle X+Y+Z=20\end{cases}</math> | ||
| − | |style="text-align:right;"|פז | + | |style="text-align:right;"|פז <big>אדם</big> שכר ג' אחים ראובן שמעון לוי שיעשו עבודתו כ' ימים מן הבקר עד הערב מאיזה מהם שיהיה ולא תשבות המלאכה<br> |
והנה אם עבד ראובן כל הימים יתן לו ה' זהובים ואם שמעון ד' ואם לוי ג‫'<br> | והנה אם עבד ראובן כל הימים יתן לו ה' זהובים ואם שמעון ד' ואם לוי ג‫'<br> | ||
והנה בין כלם עבדו הכ' שנים ימים והוא יושב ושומר עליהם כמה שעות ביום עבד כל אחד מהם וכמה חלקי שעות<br> | והנה בין כלם עבדו הכ' שנים ימים והוא יושב ושומר עליהם כמה שעות ביום עבד כל אחד מהם וכמה חלקי שעות<br> | ||
| Line 3,073: | Line 3,695: | ||
|- | |- | ||
| | | | ||
| − | : | + | :Know that Reuven is paid one zahuv for 4 days. |
| + | :<math>\scriptstyle{\color{blue}{\frac{20}{5}=4}}</math> | ||
|style="text-align:right;"|דע כי ראובן ישמש ד' ימים בזהוב אחד | |style="text-align:right;"|דע כי ראובן ישמש ד' ימים בזהוב אחד | ||
|- | |- | ||
| | | | ||
| − | + | :Shimon [is paid one zahuv] for 5 days. | |
| + | :<math>\scriptstyle{\color{blue}{\frac{20}{4}=5}}</math> | ||
|style="text-align:right;"|ושמעון ה' ימים | |style="text-align:right;"|ושמעון ה' ימים | ||
|- | |- | ||
| | | | ||
| − | + | :Levi [is paid one zahuv] for 6 days and 2-thirds of a day. | |
| + | :<math>\scriptstyle{\color{blue}{\frac{20}{3}=6+\frac{2}{3}}}</math> | ||
|style="text-align:right;"|ולוי ו' ימים וב' שלישיות יום | |style="text-align:right;"|ולוי ו' ימים וב' שלישיות יום | ||
|- | |- | ||
| | | | ||
| − | : | + | :The total is 15 days and 2-thirds. |
| + | :<math>\scriptstyle{\color{blue}{4+5+\left(6+\frac{2}{3}\right)=15+\frac{2}{3}}}</math> days | ||
|style="text-align:right;"|והנה הכל ט"ו ימים וב' שלישיות אחד | |style="text-align:right;"|והנה הכל ט"ו ימים וב' שלישיות אחד | ||
|- | |- | ||
| | | | ||
| − | : | + | :We divide 20 by this number; the result is one integer; 4 integers and a third remain. |
| − | + | |style="text-align:right;"|נחלק כ' על זה המספר ועלה אחד שלם ונשארו ד' שלמים ושלישית | |
| − | |||
| − | |style="text-align:right;"|נחלק כ' על זה המספר ועלה אחד שלם ונשארו ד' שלמים ושלישית | ||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :We convert all into thirds because of the third: |
| + | |style="text-align:right;"|והנה בעבור השלישית נשים הכל שלישיות | ||
|- | |- | ||
| | | | ||
| − | : | + | :We convert the 20 days into 60-thirds; the 15 and 2-thirds into 47-thirds; and the 4 and a third into 13. |
| − | |style="text-align:right;"|והנה | + | |style="text-align:right;"|והנה נשיב הכ' ימים ס' שלשיות והט"ו וב' שלישיו' מ"ז שלשיות והד' ושלישית י"ג |
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{ | + | :So, each one was paid one zahuv and 13 pešuṭim. |
| − | + | |style="text-align:right;"|והנה כל אחד לקח זהוב אחד וי"ג פשו' ממטבע מ"ז בזהוב | |
| − | + | |- | |
| − | + | | colspan="2"| | |
| − | + | :<math>\scriptstyle{\color{blue}{\frac{20}{15+\frac{2}{3}}=1+\frac{4+\frac{1}{3}}{15+\frac{2}{3}}=\frac{\frac{60}{3}}{\frac{47}{3}}=1+\frac{\frac{13}{3}}{\frac{47}{3}}=1+\frac{13}{47}}}</math> | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :Now, we check how much each must work, so that they complete the 20 days. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ועתה נבקש כמה חייב כל אחד לעבוד עד שישלימו הכ' ימים |
|- | |- | ||
| | | | ||
| − | : | + | :We start with Levi, who has to work 6 days and 2-thirds for the zahuv he is paid. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והנה נחל מלוי שחייב לשמש בזהוב שלקח ו' ימים וב' שלישיות |
|- | |- | ||
| | | | ||
| − | : | + | :We convert them into thirds; they are 20. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ונעשה מאלו שלשיות ויהיו כ‫' |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | :: | + | :We want to know how much he must work for the 13 pešuṭim he received: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ונבקש לדעת כמה יש לו לעבוד בעבור יג"פ שלקח |
|- | |- | ||
| | | | ||
| − | : | + | :We multiply 13 by 20; it is 260. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כפלנו י"ג על כ' היו ר"ס |
|- | |- | ||
| | | | ||
| − | : | + | :We divide it by 47; the result is 5 and 25 parts remain. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|חלקנום על מ"ז עלו ה' נשארו כ"ה חלקי‫' |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :We add 5 to 20; they are 25-thirds and 25 parts of 47. |
| − | + | |style="text-align:right;"|חברנו ה' עם כ' היו כ"ה שלשיות וכ"ה חלקי' ממ"ז | |
| − | |style="text-align:right;"| | ||
| − | |||
| − | |||
|- | |- | ||
| − | |||
| | | | ||
| + | :We divide the thirds by 3; the result is 8 integers and one remains. | ||
| + | |style="text-align:right;"|חלקנו אלו השלישיות על ג' עלו ח' שלמים ונשאר אחד | ||
|- | |- | ||
| | | | ||
| − | : | + | :We consider it as 4 hours, which are a third of a day. |
| − | + | |style="text-align:right;"|נקח לו ד' שעות שהם שלישית יום | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |style="text-align:right;"| | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | + | :We multiply 25 by 4; the result is 100. | |
| + | |style="text-align:right;"|נכפול כ"ה על ד' עלו ק‫' | ||
| + | |- | ||
| | | | ||
| + | :We divide it by 47; the result is 2 hours and 6 parts remain. | ||
| + | |style="text-align:right;"|נחלקם על מ"ז עלו ב' שעות ונשארו ו' חלקי‫' | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | :<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle\left(1+\frac{13}{47}\right)\sdot\left(6+\frac{2}{3}\right)&\scriptstyle=\left(1+\frac{13}{47}\right)\sdot\frac{20}{3}=\frac{20}{3}+\frac{\frac{13\sdot20}{47}}{3}=\frac{20}{3}+\frac{\frac{260}{47}}{3}=\frac{20}{3}+\frac{5}{3}+\frac{\frac{25}{47}}{3}=\frac{25}{3}+\frac{\frac{25}{47}}{3}\\&\scriptstyle=8+\frac{1}{3}+\frac{\frac{25}{47}}{3}=8+\frac{4}{12}+\frac{\frac{4\sdot25}{47}}{12}=8+\frac{4}{12}+\frac{\frac{100}{47}}{12}\\&\scriptstyle=8+\frac{4}{12}+\frac{2}{12}+\frac{\frac{6}{47}}{12}=8+\frac{6}{12}+\frac{\frac{6}{47}}{12}\\\end{align}}}</math> | ||
|- | |- | ||
| | | | ||
| − | : | + | :So, Levi worked 8 days, 6 hours and 6 parts [of 47]. |
| − | |style="text-align:right;"|והנה ''' | + | |style="text-align:right;"|והנה לוי עבד ח' ימים ו' שעות ו' חלקי‫' |
|- | |- | ||
| | | | ||
| − | : | + | :We want to know how much Shimon worked, who has to work 5 days, which is 15-thirds, for the zahuv he is paid. |
| − | |style="text-align:right;"|ו | + | :<math>\scriptstyle{\color{blue}{5=\frac{15}{3}}}</math> |
| + | |style="text-align:right;"|נבקש לדעת כמה עבד שמעון הוא חייב לעבוד בעבור הזהוב ה' ימים שהם ט"ו שלישיות | ||
|- | |- | ||
| | | | ||
| − | : | + | :We want to know how much he must work for the 13 pešuṭim he received: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ונבקש לדעת כמה יעבוד בעבור יג"פ שלקח |
|- | |- | ||
| | | | ||
| − | + | :We multiply 13 by 15; the result is 195. | |
| − | + | |style="text-align:right;"|נכפול י"ג על ט"ו עלו קצ"ה | |
| + | |- | ||
| | | | ||
| + | :We divide it by 47; the result is 4 and 7 parts remain. | ||
| + | |style="text-align:right;"|נחלקם על מ"ז יעלו ד' ונשאר ז' חלקי‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | :We add 4 to 15, because they are thirds; they are 19-thirds. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|נחבר הד' אל הט"ו כי שלשיות הם יהיו י"ט שלשיות |
|- | |- | ||
| | | | ||
| − | : | + | :We divide them by 3; they are 6 whole days. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|נחלקם על ג' יהיו ו' ימים שלמים |
|- | |- | ||
| | | | ||
| − | : | + | :We consider the one that remains as 4 hours. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ונקח לאחד הנשאר ד' שעו‫' |
|- | |- | ||
| | | | ||
| − | : | + | :We also multiply 7 by 4; it is 28. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|גם נכפול ז' על ד' יהיו כ"ח |
| + | |- | ||
| + | | colspan="2"| | ||
| + | :<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle\left(1+\frac{13}{47}\right)\sdot5&\scriptstyle=\left(1+\frac{13}{47}\right)\sdot\frac{15}{3}=\frac{15}{3}+\frac{\frac{13\sdot15}{47}}{3}=\frac{15}{3}+\frac{\frac{195}{47}}{3}=\frac{15}{3}+\frac{4}{3}+\frac{\frac{7}{47}}{3}=\frac{19}{3}+\frac{\frac{7}{47}}{3}\\&\scriptstyle=6+\frac{1}{3}+\frac{\frac{7}{47}}{3}=6+\frac{4}{12}+\frac{\frac{4\sdot7}{47}}{12}=6+\frac{4}{12}+\frac{\frac{28}{47}}{12}\\\end{align}}}</math> | ||
|- | |- | ||
| | | | ||
| − | : | + | :So, Shimon worked 6 days, 4 hours and 28 parts [of 47]. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והנה הם ו' ימים וד' שעות וכ"ח חלקי' וככה עבד שמעון |
|- | |- | ||
| | | | ||
| − | ::{| | + | :We want to know how much Reuven must work: he must work 4 days for the one zahuv, which are 12 thirds. |
| + | :<math>\scriptstyle{\color{blue}{4=\frac{12}{3}}}</math> | ||
| + | |style="text-align:right;"|נבקש לדעת כמה עבד ראובן והנה עבד בשביל הזהוב ד' ימים שהם י"ב שלשיות | ||
|- | |- | ||
| | | | ||
| − | + | :We extract the ratio for the 13 pešuṭim he received: | |
| + | |style="text-align:right;"|ונעשה בעבור היג"פ שלקח הערך | ||
|- | |- | ||
| − | | | + | | |
| + | :We multiply 13 by 12; the result is 156. | ||
| + | |style="text-align:right;"|<s>נפ</s> נכפול י"ג על י"ב עלו קנ"ו | ||
|- | |- | ||
| − | | | + | | |
| − | | | + | :We divide it by 47; the result is 3 and 15 parts remain. |
| + | |style="text-align:right;"|נחלקם על מ"ז עלו ג' ונשארו ט"ו חלקים | ||
|- | |- | ||
| − | |||
| | | | ||
| − | + | :We add 3 to the 12 we have; they are 15-thirds. | |
| + | |style="text-align:right;"|חברנו אלו הג' עם הי"ב שהיו לנו היו ט"ו והם שלשיות | ||
|- | |- | ||
| | | | ||
| − | + | :So, he worked 5 days. | |
| + | |style="text-align:right;"|והנה עבד ה' ימים | ||
|- | |- | ||
| − | | | + | | |
| + | :We also multiply the 15 parts by 4; the result is 60. | ||
| + | |style="text-align:right;"|גם הט"ו חלקי' נכפול על ד' עלו ס‫' | ||
|- | |- | ||
| − | | | + | | |
| − | + | :We divide it by 47; the result is one hour and 13 parts of an hour remain in which Reuven worked. | |
| + | |style="text-align:right;"|נחלקם על מ"ז עלתה שעה אחת ונשארו י"ג חלקי' משעה שעבד ראובן | ||
|- | |- | ||
| − | |} | + | | colspan="2"| |
| + | :<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle\left(1+\frac{13}{47}\right)\sdot4&\scriptstyle=\left(1+\frac{13}{47}\right)\sdot\frac{12}{3}=\frac{12}{3}+\frac{\frac{13\sdot12}{47}}{3}=\frac{12}{3}+\frac{\frac{156}{47}}{3}=\frac{12}{3}+\frac{3}{3}+\frac{\frac{15}{47}}{3}=\frac{15}{3}+\frac{\frac{15}{47}}{3}\\&\scriptstyle=5+\frac{\frac{15}{47}}{3}=5+\frac{\frac{4\sdot15}{47}}{12}=5+\frac{\frac{60}{47}}{12}=5+\frac{1}{12}+\frac{\frac{13}{47}}{12}\\\end{align}}}</math> | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Check:'''</span> When you add up these parts, the result is one hour, no more and no less. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכאשר תחבר אלה החלקי' יעלה מהם שעה אחת בלי תוספת ומגרעת |
|- | |- | ||
| | | | ||
| − | : | + | :When you add this hour to the mentioned hours, they are 12 hours, which is one day. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכאשר תחבר שעה זו לשעות הנזכרות יהיו י"ב שעות שהוא יום אחד |
|- | |- | ||
| | | | ||
| − | :: | + | :When you add this day to the mentioned days, they are 20 days, no more and no less. |
| + | |style="text-align:right;"|וכאשר תחבר היום לימים הנז' יהיו כ' ימים בלי תוספת ומגרעת וכה"ל | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | :<math>\scriptstyle{\color{blue}{\left(8+\frac{6}{12}+\frac{\frac{6}{47}}{12}\right)+\left(6+\frac{4}{12}+\frac{\frac{28}{47}}{12}\right)+\left(5+\frac{1}{12}+\frac{\frac{13}{47}}{12}\right)=20}}</math> | ||
|- | |- | ||
| | | | ||
| − | + | ||
| + | === <span style=color:green>Divide a Quantity Problem - Proportional Division – Inheritance</span> === | ||
| + | |||
| + | | | ||
|- | |- | ||
| − | | | + | | |
| + | :{{#annot:inheritance|645|xX25}}88) Jacob died. | ||
| + | :Reuven issued a deed with two witnesses, according to which his father Jacob has given him all the property he had and instructed so in case of death. | ||
| + | :His son Shimon issued a deed as well according to which half of his property should be granted to him. | ||
| + | :His son Levi also issued a deed according to which a third of his property should be given to him. | ||
| + | :His son Yehudah too issued a deed according to which a quarter of his property should be granted to him. | ||
| + | :All of them are writing this in Jerusalem in the same day, the same time, the same hour | ||
| + | |style="text-align:right;"|פח <big>שאלה</big> יעקב מת<br> | ||
| + | והוציא ראובן שטר בשני עדים כשרים שנתן לו יעקב אביו כל ‫<ref>97r</ref>הממון שהיה לו וציוה כן מחמת מיתה<br> | ||
| + | גם הוציא שמעון בנו שטר שיינתן לו חצי ממונו<br> | ||
| + | גם הוציא לוי שטר שינתן לו שליש ממונו<br> | ||
| + | גם הוציא יהודה שטר שינתן לו רביע ממונו<br> | ||
| + | וכולם ביום אחד וזמן אחד ושעה אחת ברושלים שכותבים בו שעות{{#annotend:xX25}} | ||
| + | |- | ||
| + | |<span style=color:green>'''Three methods to divide the property between the four sons each according to his relative portion:'''</span> | ||
| + | | | ||
|- | |- | ||
| − | | | + | | |
| − | | | + | *'''The sages of Israel''' divide it according to the request of each. |
| + | |style="text-align:right;"|והנה ''חכמי ישראל'' מחלקים אותו על דרך בקשת כל אחד | ||
|- | |- | ||
| − | |||
| | | | ||
| − | + | *'''The Gentile sages''' - according to the ratio of each share. | |
| + | |style="text-align:right;"|ו''חכמי הגוים'' על דרך ערך הממון של כל אחד | ||
|- | |- | ||
| | | | ||
| − | {|style=" | + | *'''The arithmeticians''' consider the property as a whole, so when you add to it its half, its third, and its quarter, the total is two and half a sixth. |
| + | :<math>\scriptstyle X+\frac{1}{2}X+\frac{1}{3}X+\frac{1}{4}X=120</math> | ||
| + | :<math>\scriptstyle{\color{blue}{1+\frac{1}{2}+\frac{1}{3}+\frac{1}{4}=2+\left(\frac{1}{2}\sdot\frac{1}{6}\right)}}</math> | ||
| + | |style="text-align:right;"|ו''חכמי החשבון'' יחשבו כי הממון היה אחד וכאשר תחבר אליו חציתו ושלשיתו ורבעיתו יהיו הכל שנים וחצי ששית | ||
|- | |- | ||
| − | | | + | | |
| + | :<span style=color:green>'''False Position:'''</span> We suppose the whole is 60, which has all the mentioned parts; their sum is 125. | ||
| + | :<math>\scriptstyle{\color{blue}{\left[2+\left(\frac{1}{2}\sdot\frac{1}{6}\right)\right]\sdot60=125}}</math> | ||
| + | |style="text-align:right;"|והנה נשים האחד שלם ס' שיש לו כל החלקי' הנז' ויהיו בין הכל קכ"ה | ||
|- | |- | ||
| − | | | + | | |
| − | + | :<span style=color:green>'''Another false position:'''</span> Or, we suppose the whole is 12, and the [sum of the] mentioned fractions is 13. | |
| + | :<math>\scriptstyle{\color{blue}{\left[2+\left(\frac{1}{2}\sdot\frac{1}{6}\right)\right]\sdot12=12+13}}</math> | ||
| + | |style="text-align:right;"|או נשים האחד שלם י"ב והשברים הנז' י"ג | ||
|- | |- | ||
| − | | | + | | |
| + | :The result, for whichever you take, will be the same. | ||
| + | |style="text-align:right;"|ושוה יצא המספר באחרונה אי זה מהם שתקח | ||
|- | |- | ||
| | | | ||
| − | : | + | :Now, we look how much Reuven will take according to the value of his share: we extract the ratio from 60, as he ask for the whole property. We suppose the property is 10 dinar that are 120 pešuṭim. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ועתה נבקש כמה יקח ראובן לפי ערך ממונו ונעשה הערך ככה על דרך ס' כי הוא מבקש כל הממון ונעשה כי הממון י' די' שהם ק"כ פ‫' |
|- | |- | ||
| | | | ||
| − | : | + | :This is the diagram of the share that Reuven takes: |
| − | |style="text-align:right;"|וזה צורת | + | |style="text-align:right;"|וזה צורת ערך הממון שיקח ראובן |
|- | |- | ||
| | | | ||
| − | + | :{|class="wikitable" | |
|- | |- | ||
| | | | ||
{|style="color: blue; text-align:center;" | {|style="color: blue; text-align:center;" | ||
|- | |- | ||
| − | |0|| | + | |0||60 |
|- | |- | ||
|120||125 | |120||125 | ||
| Line 3,301: | Line 3,952: | ||
{|style="margin-left: auto; margin-right: 0px; text-align:center;" | {|style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
|- | |- | ||
| − | |‫0||‫ | + | |‫0||‫0ו |
|- | |- | ||
|‫0בא||הבא | |‫0בא||הבא | ||
| Line 3,309: | Line 3,960: | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> We multiply the extremes; the result is 7200. We divide it by 125; the result is 57 pešuṭim and 75 parts and this is Reuven's share. | |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{\frac{60\sdot120}{125}=\frac{7200}{125}=57+\frac{75}{125}}}</math> |
| + | |style="text-align:right;"|כפלנו הקצוות עלו ז' אלפי' ור‫'<br> | ||
| + | חלקנום על קכ"ה עלו נ"ז פשו' וע"ה חלקי' וזה חלק ראובן | ||
|- | |- | ||
| | | | ||
| − | : | + | :This is the diagram of Shimon's share: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וזה צורת ערך שמעון |
|- | |- | ||
| | | | ||
| − | + | :{|class="wikitable" | |
|- | |- | ||
| | | | ||
{|style="color: blue; text-align:center;" | {|style="color: blue; text-align:center;" | ||
|- | |- | ||
| − | |0|| | + | |0||30 |
|- | |- | ||
|120||125 | |120||125 | ||
| Line 3,334: | Line 3,987: | ||
{|style="margin-left: auto; margin-right: 0px; text-align:center;" | {|style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
|- | |- | ||
| − | |‫0|| | + | |‫0||‫0ג |
|- | |- | ||
|‫0בא||הבא | |‫0בא||הבא | ||
| Line 3,342: | Line 3,995: | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> We multiply the extremes and divide according to the rule. | |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{\frac{30\sdot120}{125}}}</math> |
| + | |style="text-align:right;"|נכפול הקצוות ונחלק כמשפט | ||
|- | |- | ||
| | | | ||
| − | + | :This is the diagram of Levi's share: | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וזה צורת חלק לוי |
|- | |- | ||
| | | | ||
| − | : | + | :{|class="wikitable" |
| − | | | ||
|- | |- | ||
| | | | ||
| − | + | {|style="color: blue; text-align:center;" | |
| − | |style="text-align: | + | |- |
| + | |0||20 | ||
| + | |- | ||
| + | |120||125 | ||
| + | |} | ||
|- | |- | ||
| + | |} | ||
| | | | ||
| − | + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | |
| − | |||
|- | |- | ||
| | | | ||
| − | + | {|style="margin-left: auto; margin-right: 0px; text-align:center;" | |
| − | |style="text-align: | ||
|- | |- | ||
| − | | | + | |‫0||‫0ב |
| − | |||
| − | | | ||
|- | |- | ||
| − | | | + | |‫0בא||הבא |
| − | + | |} | |
| − | |||
|- | |- | ||
| − | | | + | |} |
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :<span style=color:green>'''Rule of Three:'''</span> We multiply and divide according to the rule. |
| − | :: | + | :<math>\scriptstyle{\color{blue}{\frac{20\sdot120}{125}}}</math> |
| − | : | + | |style="text-align:right;"|ונכפול ונחלק כמשפט |
| − | |style="text-align:right;"| | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :This is the diagram of Yehudah's share: |
| − | + | |style="text-align:right;"|וזו צורת חלק יהודה | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | : | + | :{|class="wikitable" |
| − | | | ||
|- | |- | ||
| | | | ||
| − | + | {|style="color: blue; text-align:center;" | |
| − | |||
| − | |||
| − | |style="text-align: | ||
|- | |- | ||
| − | | | + | |0||15 |
| − | |||
| − | | | ||
|- | |- | ||
| + | |120||125 | ||
|} | |} | ||
| − | |||
|- | |- | ||
| + | |} | ||
| | | | ||
| − | + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | |
| − | + | |- | |
| − | |||
| | | | ||
| + | {|style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | |‫0||הא | ||
|- | |- | ||
| − | | | + | |‫0בא||הבא |
| − | | | + | |} |
|- | |- | ||
| − | | | + | |} |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :<span style=color:green>'''Rule of Three:'''</span> We multiply and divide according to the rule. |
| − | | | + | :<math>\scriptstyle{\color{blue}{\frac{15\sdot120}{125}}}</math> |
| + | |style="text-align:right;"|ונכפול ונחלק כמשפט | ||
|- | |- | ||
| | | | ||
| − | + | *Another shorter method [according to the '''Gentile sages''']: | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ענין אחר בדרך קצרה |
|- | |- | ||
| | | | ||
| − | : | + | :Shimon takes a half of Reuven's share. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|יקח שמעון חצי חלק ראובן |
|- | |- | ||
| | | | ||
| − | : | + | :Levi takes a third of Reuven's share. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ולוי יקח שליש חלק ראובן |
|- | |- | ||
| | | | ||
| − | : | + | :Yehudah takes a quarter of Reuven's share. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ויהודה יקח רביע חלק ראובן |
|- | |- | ||
| − | |||
| | | | ||
| + | :When you sum up all these fractions and the integers, they are 120 pešuṭim that are 10 dinar. | ||
| + | |style="text-align:right;"|וכאשר תחבר אלו כל החלקי' והשלמים יהיו ק"כ פ' שהם י' די‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | *According to the method of the '''sages of Israel''': |
| − | + | |style="text-align:right;"|ועל דרך ''חכמי ישראל'' | |
| − | |style="text-align:right;"| | ||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :The three older brothers say to Yehudah: you are only appealing about 30 pešuṭim and the appeal of each of us is the same about them, so take 7 and a half, which is a quarter and leave us. |
| − | :< | + | :<math>\scriptstyle{\color{blue}{\frac{1}{4}\sdot30=7+\frac{1}{2}}}</math> |
| − | | | + | |style="text-align:right;"|יאמר הג' אחים הגדולים ליהודה אין אתה מערער רק על ל"פ וערעור כל אחד ממנו שוה בהם קח ז' וחצי שהוא הרבעית ולך מעמנו |
|- | |- | ||
| | | | ||
| − | : | + | :Each of the three brothers takes the same also. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכמו כן יקח כל אחד מהג' אחים |
|- | |- | ||
| | | | ||
| − | :: | + | :Then, Reuven says to Levi: you are only appealing about 40 pešuṭim and you have already taken your share from the 30 the four of us shared, so take a third of 10, which is a quarter of 40 and leave us. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ועוד יאמר ראובן ללוי אין אתה מערער רק על מ"פ וכבר לקחת חלקך מהל' שהארבעתינו חלקנו עליו<br> |
| + | קח אתה שלישית י' שהוא רבעית מ' ולך מעמנו | ||
|- | |- | ||
| | | | ||
| − | : | + | :Hence, Levi's share is 10 pešuṭim and 5-sixths, because the half from the 7 and a half he already took is 3-sixths and the third from the 10 are 2-sixths, so they are 5-sixths |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והנה חלק לוי י' וה' ששיות פשוטי כי החצי מן הז' וחצי שלקח כבר הם ג' ששיות ושליש אחד מן הי' הם ב' ששיות הרי ה' ששיות |
|- | |- | ||
| − | | | + | | colspan="2"| |
| − | : | + | :<math>\scriptstyle{\color{blue}{\left(\frac{1}{4}\sdot30\right)+\left[\frac{1}{3}\sdot\left(\frac{1}{4}\sdot40\right)\right]=\left(7+\frac{1}{2}\right)+\left(\frac{1}{3}\sdot10\right)=\left(7+\frac{3}{6}\right)+\left(3+\frac{2}{6}\right)=10+\frac{5}{6}}}</math> |
| − | |||
|- | |- | ||
| | | | ||
| − | :: | + | :Reuven also says to Shimon: you are only appealing about one half, which is 60; all the other half is mine; and you have already taken your share from the 40, so take half of the appeal that remains between you and me, and leave me. |
| − | | | + | |style="text-align:right;"|גם ראובן יאמ' לשמעון אין אתה מערער רק על חצי האחד שהוא ס' וחצי האחר כולו שלי וכבר לקחת חלק מהמ' והנה שנשאר בינך ובני הערעור קח חציים ולך מעלי |
|- | |- | ||
| | | | ||
| − | : | + | :So, Shimon's share is 20 pešuṭim and 5-sixths. |
| − | ::<math>\scriptstyle{\color{blue}{ | + | |style="text-align:right;"|והנה חלק שמעון כ' וה' ששיות פשוט |
| − | + | |- | |
| + | | colspan="2"| | ||
| + | :<math>\scriptstyle{\color{blue}{\left(\frac{1}{4}\sdot30\right)+\left[\frac{1}{3}\sdot\left(\frac{1}{4}\sdot40\right)\right]+\left[\frac{1}{2}\sdot\left(\frac{1}{3}\sdot60\right)\right]=20+\frac{5}{6}}}</math> | ||
|- | |- | ||
| | | | ||
| − | : | + | :Reuven's share is 80 pešuṭim and 5-sixths. |
| − | + | |style="text-align:right;"|וחלק ראובן פ' וה' ששיות פשוט | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| − | | | + | | colspan="2"| |
| − | + | :<math>\scriptstyle{\color{blue}{\left(\frac{1}{4}\sdot30\right)+\left[\frac{1}{3}\sdot\left(\frac{1}{4}\sdot40\right)\right]+\left[\frac{1}{2}\sdot\left(\frac{1}{3}\sdot60\right)\right]+\left(\frac{1}{2}\sdot120\right)=80+\frac{5}{6}}}</math> | |
|- | |- | ||
| | | | ||
| − | : | + | :When you sum up all these shares, they are 10 dinar. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכאשר תחבר אלו החלקי' יהיו י' די' וכה"ל |
|- | |- | ||
| − | | | + | |} |
| − | + | {| | |
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | + | ||
| − | + | == <span style=color:green>Geometrical Problems</span> == | |
| − | + | ||
| | | | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | + | === <span style=color:green>Triangulation Problem - Two towers</span> === | |
| − | + | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| | | | ||
|- | |- | ||
| | | | ||
| − | :{{#annot: | + | :{{#annot:two birds|655|YOsy}}89) two towers, one is 15 cubits tall and the other is 12 cubits tall. |
| − | : | + | :There are two pigeons there, [one pigeon at the top of each tower], and between them a spring. |
| − | : | + | :[The pigeons] arrive to the spring at the same moment. |
| − | |style="text-align:right;"| | + | :How far are the towers from each other and where is the place of the spring? |
| − | + | :<math>\scriptstyle15^2+a^2=12^2+b^2</math> | |
| + | |style="text-align:right;"|פט <big>ב</big> מגדלים אחד גבוה ט"ו אמות ואחד גבוה י"ב אמות<br> | ||
| + | ושם ב' יונים ומעיין אחד ביניהם ומגיעים ברגע אחד במעיין<br> | ||
| + | כמה מרוחקים המגדלים זה מזה ואנה מקום המעיין{{#annotend:YOsy}} | ||
|- | |- | ||
| | | | ||
| − | :[[File:Kelalim ba-Mispar | + | :[[File:Kelalim ba-Mispar 89.png|thumb|200px|left]] |
| − | |||
| | | | ||
|- | |- | ||
| | | | ||
| − | : | + | :Suppose the distance between them is the same as the measure of both heights, i.e. 12 plus 15, which is 27. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{12+15=27}}</math> |
| + | |style="width:45%;text-align:right;"|שים הרוחק שבניהם כמדת גובה שתיהם דהיינו י"ב וט"ו שהם כ"ז | ||
|- | |- | ||
| | | | ||
| − | : | + | :Divide 27 into 12 and 15. |
| − | + | :<math>\scriptstyle{\color{blue}{27=12+15}}</math> | |
| − | + | |style="text-align:right;"|וחלק כ"ז לי"ב ולט"ו | |
| − | |style="text-align:right;"| | ||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :Give the 15 to the tower that is 12 cubits high. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ושים הט"ו אצל המגדל שהוא גבוה י"ב |
|- | |- | ||
| | | | ||
| − | : | + | :Give the 12 to the tower that is 15 cubits high. |
| − | + | |style="text-align:right;"|ושים הי"ב אצל המגדל שהוא גבוה ט"ו | |
| − | |||
| − | |style="text-align:right;"| | ||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :The place of the spring is 12-15 cubits from the bases [of the towers]. |
| − | + | |style="text-align:right;"|ובגבול הט"ו והי"ב מן התושבת שם המעיין וכה"ל | |
| − | |||
| − | |style="text-align:right;"| | ||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | === <span style=color:green>Rectangle</span> === |
| − | + | ||
| − | |||
| − | |||
| | | | ||
|- | |- | ||
| + | !<span style=color:green>Figure Problem - Side; Diagonal; Area – Rectangle</span> | ||
| | | | ||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :{{#annot:rectangle|679,683,680|9HVg}}90) Question: a rectangle, its diagonal together with its one side are 18, and its other side is 6. |
| − | |style="text-align:right;"| | + | :How much are its area, its diagonal and its side that is summed with the diagonal? |
| + | |style="text-align:right;"|צ <big>שאלה</big> {{#annot:term|591,1525|euTK}}מרובע ארוך{{#annotend:euTK}} אשר אלכסונו עם צלעו האחד י"ח וצלעו השנית ו‫'<br> | ||
| + | כמה הוא ריבועו ואלכסונו וצלעו המנויה עם האלכסון{{#annotend:9HVg}} | ||
|- | |- | ||
| − | | | + | | |
| + | :[[File:Kelalim ba-Mispar 90.png|thumb|75px|left]] | ||
| + | :<span style=color:red>[this figure does not appear in the text]</span> | ||
| | | | ||
|- | |- | ||
| | | | ||
| − | : | + | :The one who answers this question squares the known side, which is 6; its square is 36. |
| − | + | |style="text-align:right;"|והמשיב על השאלה הזאת ‫<ref>97v</ref>ירבע את הצלע הידועה והוא ו' ויהיה רביעו ל"ו | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | : | + | :He divides it by the sum of the diagonal and the second side, which is 18; the quotient is 2. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ויחלקם על מניין האלכסון והצלע השנית שהוא י"ח ותהיה החלוקה ב‫' |
|- | |- | ||
| − | |||
| | | | ||
| + | :He adds 2 to 18; it is 20. | ||
| + | |style="text-align:right;"|ויוסיף ב' על י"ח ויהיו כ‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | :So, he knows that half 20 is the diagonal. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וידע כי מחצית כ' הוא האלכסון |
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{2}\sdot\left(18+\frac{6^2}{18}\right)=\frac{1}{2}\sdot\left(18+\frac{36}{18}\right)=\frac{1}{2}\sdot\left(18+2\right)=\frac{1}{2}\sdot20}}</math> | ||
|- | |- | ||
| | | | ||
| − | :the | + | :The remainder from 18 is the second side; it is 8. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{18-10=8}}</math> |
| + | |style="text-align:right;"|והנשאר מי"ח הוא הצלע השנית והוא ח‫' | ||
|- | |- | ||
| | | | ||
| − | :the | + | :Their product, 8 by 6, is the area. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{8\times6}}</math> |
| + | |style="text-align:right;"|ורבועם ח' בו' והוא התשבורת | ||
|- | |- | ||
| | | | ||
| − | + | === <span style=color:green>Quadrangle</span> === | |
| − | + | ||
| − | |||
| | | | ||
| − | |||
|- | |- | ||
| + | !<span style=color:green>Figure Problem - Area - Quadrangle</span> | ||
| | | | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :{{#annot:quadrangle|680|wUZ0}}91) A quadrangle whose sides are not equal - its length is not equal to its breadth, the one length is not equal to the other length, and the one breadth is also not equal to the other breadth. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|צא <big>הרי</big> שיש לפניך א' מרובע שאין ארכו כרחבו ואינו שוה האורך האחד לאורך השני וגם רוחב האחד אינו שוה לרוחב השני{{#annotend:wUZ0}} |
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | + | :Add up the lengths, divide [the sum] in half and keep its half. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|{{#annot:term|178,1810|LVqT}}צרף{{#annotend:LVqT}} האורך וחלקהו לחצי ותפוש חציו |
|- | |- | ||
| | | | ||
| − | : | + | :Add up the breadth with the breadth, and keep its half. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וצרף הרוחב עם הרוחב ותפוש חציו |
|- | |- | ||
| | | | ||
| − | : | + | :Multiply half the sum of the two lengths by half the sum of the two breadths; its area is as their product. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ותרבה החצי משני הארכים על החצי משני הרחבים וכפי מספר אותם הרבויים יהיו מספר שבריו |
|- | |- | ||
| − | | | + | | colspan="2"| |
| − | + | :<math>\scriptstyle\scriptstyle{\color{OliveGreen}{\left[\frac{1}{2}\sdot\left(\rm{length_1+length_2}\right)\right]\sdot\left[\frac{1}{2}\sdot\left(\rm{breadth_1+breadth_2}\right)\right]}}</math> | |
|- | |- | ||
| | | | ||
| − | + | :<span style=color:red>[The author does not note that this formula is not accurate in the general case]</span> | |
| − | |||
| − | |||
| | | | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | + | *{{#annot:quadrangle|680|1rAc}}Example: its one length is 4, and its other length is 6 its one breadth is 5, and its other breadth is 3 | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והמשל בזה הרי שהיה מקום אחד ארכו האחד ד' ואורכו השני ו' ורחבו האחד ה' ורחבו השני ג' כזה{{#annotend:1rAc}} |
|- | |- | ||
| | | | ||
| − | :: | + | ::[[File:Kelalim ba-Mispar 91.png|thumb|100px|left]] |
| − | | | + | |[[File:כללים במספר - צא.png|thumb|100px]] |
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :Add the length to the length and say: 6 plus 4 is 10. Take its half; it is 5. Keep it. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|צרף האורך עם האורך ואמ' ו' וד' הרי י' תפוס חציו הרי ה' ויהיה ד' ארכו ו' לך למשמר |
|- | |- | ||
| | | | ||
| − | + | :Then add the breadth to the breadth and say: 3 plus 5 is 8. Take its half; it is 4. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אחרי כן צרף הרוחב עם הרוחב ואמור ג' וה' הרי ח' תפוס חציו שהוא ד' רחבו ה‫' |
|- | |- | ||
| | | | ||
| − | :: | + | :Multiply it by 5, which is half the sum of the two lengths; say: 4 by 5 is 20. So, its area is 20. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ותרבה אותו עם הה' שהוא מחצית ב' האורכים ואמור ד' פעמ' ה' הם כ' הרי ש{{#annot:term|816,1242|1sqc}}מידת שבריו{{#annotend:1sqc}} הם כ' וכה"ל |
|- | |- | ||
| − | | | + | |colspan=2| |
| − | : | + | :<math>\scriptstyle{\color{blue}{\left[\frac{1}{2}\sdot\left(4+6\right)\right]\sdot\left[\frac{1}{2}\sdot\left(5+3\right)\right]=\left(\frac{1}{2}\sdot10\right)\sdot\left(\frac{1}{2}\sdot8\right)=5\sdot4=20}}</math> |
| − | |||
|- | |- | ||
| | | | ||
| − | + | ||
| − | + | === <span style=color:green>Triangulation Problem - Tree leaning against a wall</span> === | |
| − | + | ||
| − | |||
| | | | ||
|- | |- | ||
| | | | ||
| − | : | + | :{{#annot:tree|655|wifm}}92) A tree 30 cubits high was standing straight next to a wall. |
| − | |style="text-align:right;"| | + | :Then it was withdrawn from the bottom [of the wall] by 10 cubits [so that its top is leaning against the wall]. |
| + | :By how much was the top of the tree brought down from its original place? | ||
| + | |style="text-align:right;"|צב <big>הרי</big> שעץ אחד גבוה ל' אמות וזקוף בצד חומה אחת והוסר מלמטה י' אמות<br> | ||
| + | נרצה לידע מזה כמה הורד ראש העץ ממקום שהיה{{#annotend:wifm}} | ||
|- | |- | ||
| | | | ||
| − | + | :[[File:Kelalim ba-Mispar 92.png|thumb|100px|left]]<br> | |
| − | | | + | :<span style=color:red>[this figure does not appear in the text]</span> |
| + | | | ||
|- | |- | ||
| | | | ||
| − | : | + | :You know that from the top of the tree to the ground and from the wall to the base of the tree is a right angle. |
| − | <span style=color: | + | |style="text-align:right;"|אתה יודע כי החומה דהיינו מראש העץ עד הקרקע והקרקע דהיינו מן החומה עד סוף העץ הם שני זוויות על זוית נצבה |
| + | |- | ||
| + | | | ||
| + | :<span style=color:green>'''Pythagorean Theorem:'''</span> | ||
| | | | ||
|- | |- | ||
| | | | ||
| − | : | + | :The hypotenuse is the tree, which is 30 cubits. Its square is 900. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וה{{#annot:term|1607,1878|QiEw}}אלכסון{{#annotend:QiEw}} שלהם הוא העץ שהוא ל' אמות ושברי מרובע שיוצא ממנו הם תת"ק |
|- | |- | ||
| | | | ||
| − | : | + | :Then, square the line of the ground, which is 10; its square is 100. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואחר כן תרבע קו הקרקע שהוא י' ושבריו הם ק‫' |
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | :Subtract it from 900; 800 remains. |
| + | |style="text-align:right;"|והוציאם מן התת"ק ישארו ת"ת | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | :Extract the root and it is the length of the wall; it is slightly more than 28 and a quarter. |
| + | |style="text-align:right;"|ומצא השורש והוא קו החומה ויהיה מעט יותר מכ"ח ורביע | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{30^2-10^2}=\sqrt{900-100}=\sqrt{800}\approx28+\frac{1}{4}}}</math> | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | :Subtract the length of the wall from 30 when the tree was upright; a little less than 1 and 3-quarters remain and this is [the length of the part of] the tree that was brought down. |
| + | :<math>\scriptstyle{\color{blue}{30-\left(28+\frac{1}{4}\right)=1+\frac{3}{4}}}</math> | ||
| + | |style="text-align:right;"|והוציאם מן הל' שהיה קו החומה כשהיה העץ זקוף ישארו מעט פחות מא' וג' רבעי' וכן הורד העץ | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | :Sometimes the result is exact. |
| + | |style="text-align:right;"|ולפעמי' יבא בכיוון וכה"ל | ||
|- | |- | ||
| | | | ||
| − | + | :{{#annot:tree|655|aP4N}}93) A tree 10 cubits high was standing straight next to a wall. | |
| − | |style="text-align:right;"| | + | :Then it was withdrawn from the bottom [of the wall] by 6 cubits [so that its top is leaning against the wall]. |
| + | :By how much was the top of the tree brought down from its original place? | ||
| + | |style="text-align:right;"|צג <big>וכן</big> אם היה עץ אחד גבוה י' אמות וזקוף בצד חומה והוסר מלמטה ו' אמות<br> | ||
| + | כמה הורד ראש העץ ממקום שהיה{{#annotend:aP4N}} | ||
|- | |- | ||
| | | | ||
| − | : | + | :<span style=color:green>'''Pythagorean Theorem:'''</span> |
| − | <span style=color: | ||
| | | | ||
|- | |- | ||
| | | | ||
| − | ::the | + | :Do as we did above: square the length of the tree, it is 100. |
| − | + | |style="text-align:right;"|עשה כמו שעשינו למעלה ותרבע מדת העץ והם ק‫' | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | : | + | :Then, square the 6 cubits that were brought down; it is 36. |
| − | + | |style="text-align:right;"|אחר כן תרבע הו' אמות שהורד מלמטה והם ל"ו | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | : | + | :Subtract it from 100; 64 remains. |
| − | + | |style="text-align:right;"|והוציאם מן הק' ישארו ס"ד | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | : | + | :Extract the root, meaning 8, and it is the length of the wall. |
| − | + | |style="text-align:right;"|ומצא השורש דהיינו ח' והוא קו החומה | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| − | | | + | |colspan=2| |
| − | :<math>\scriptstyle\ | + | :<math>\scriptstyle{\color{blue}{\sqrt{10^2-6^2}=\sqrt{100-36}=\sqrt{64}=8}}</math> |
| − | |||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"|'' | + | :Subtract the length of the wall from 10 when the tree was upright; you are left with [the length of the part of] the tree that was brought down, meaning 2 cubits. |
| + | :<math>\scriptstyle{\color{blue}{10-8=2}}</math> | ||
| + | |style="text-align:right;"|והוציאם מן הי' שהיה קו החומה כשהיה העץ זקוף ומה שישאר בידך הורד העץ דהיינו ב' אמות | ||
|- | |- | ||
| − | |||
| | | | ||
| + | :Now, the result is exact. | ||
| + | |style="text-align:right;"|ועתה בא בכיוון וכה"ל | ||
|- | |- | ||
| | | | ||
| − | + | ||
| − | + | === <span style=color:green>Transformation Problems</span> === | |
| − | + | ||
| − | + | | | |
|- | |- | ||
| + | !<span style=color:green>Square to Circle</span> | ||
| | | | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :{{#annot:square to circle|2583|uGlf}}94) A square, its area is 38 and a half and you want to create a circle from it, so that its area is the same as the area of the square. |
| − | + | |style="text-align:right;"|צד <big>מרובע</big> שוה צלעות ושבריו ל"ח וחצי ותרצה לעשות מהם עגול שיהיו שבריו כשברי זה המרובע{{#annotend:uGlf}} | |
| − | |style="text-align:right;"| | ||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |colspan=2| |
| − | :<math>\scriptstyle\rm{ | + | :<math>\scriptstyle\begin{cases}\scriptstyle Area_{\square}=38+\frac{1}{2}\\\scriptstyle Area_{\bigcirc}=Area_{\square}\\\scriptstyle\rm{Perimeter_{\bigcirc}}=\sqrt{4\sdot\left(3+\frac{1}{7}\right)\sdot\rm{Area_{\bigcirc}}}\end{cases}</math> |
| − | |||
|- | |- | ||
| − | |||
| | | | ||
| + | :Multiply 38 and a half by 12 and 4-sevenths; the result is 484. | ||
| + | |style="text-align:right;"|תרבה ל"ח וחצי על י"ב וד' שבעיות עולים תפ"ד | ||
|- | |- | ||
| | | | ||
| − | : | + | :Extract the root of 484; it is 22 and it is the perimeter of the circle, whose area is the same as the area of the square, whose area is 38 and a half. |
| − | + | |style="text-align:right;"|ותמצא השורש מתפ"ד והם כ"ב והוא יהיה מספר העגול יהיו שבריו כ{{#annot:term|816,1242|lZxh}}שבר{{#annotend:lZxh}} המרובע שמספר שבריו ל"ח וחצי וכה"ל | |
| − | |style="text-align:right;"| | ||
| − | |||
|- | |- | ||
| − | | | + | |colspan=2| |
| − | :[ | + | :<math>\scriptstyle{\color{blue}{\sqrt{\left(38+\frac{1}{2}\right)\sdot\left[4\sdot\left(3+\frac{1}{7}\right)\right]}=\sqrt{\left(38+\frac{1}{2}\right)\sdot\left(12+\frac{4}{7}\right)}=\sqrt{484}=22}}</math> |
| − | |||
|- | |- | ||
| + | !<span style=color:green>Circle to Square</span> | ||
| | | | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | :: | + | :{{#annot:circle to square|2583|fyX5}}95) A circle, its area is 100, and you want to create a square from it. |
| − | + | |style="text-align:right;"|צה <big>עגול</big> שבריו ק' ותרצה לעשות ממנו מרובע שוה צלעות{{#annotend:fyX5}} | |
| − | + | |- | |
| + | |colspan=2| | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle\rm{Area_{\bigcirc}}=100\\\scriptstyle \rm{Area_{\bigcirc}=Area_{\square}}\\\scriptstyle\rm{Side_{\square}=\sqrt{Area_{\square}}}\end{cases}</math> | ||
|- | |- | ||
| | | | ||
| − | :: | + | :Extract the root of 100; it is 10 and it is the size of each of its sides. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{\sqrt{100}=10}}</math> |
| + | |style="text-align:right;"|תפוס השורש מק' והוא י' והוא יהיה מדת כל צלע מצלעו וכה"ל | ||
|- | |- | ||
| | | | ||
| − | + | ||
| − | | | + | === <span style=color:green>Volume</span> === |
| + | |||
| + | | | ||
|- | |- | ||
| | | | ||
| − | + | ==== <span style=color:green>Sphere</span> ==== | |
| − | + | ||
| − | + | | | |
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :96) The volume of the sphere. |
| − | + | |style="text-align:right;"|‫<ref>98r</ref>צו <big>{{#annot:term|2219,1244|Lrs9}}תשבורת{{#annotend:Lrs9}}</big> ה{{#annot:term|1098,1892|MkFQ}}כדור{{#annotend:MkFQ}} | |
| − | |style="text-align:right;"| | ||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | :: | + | :As the geometricians stated, it is that you square the diameter of the sphere, then multiply this square 3 times and a seventh; you receive the surface of the sphere. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle\left(2r\right)^2\sdot\left(3+\frac{1}{7}\right)</math> |
| + | |style="text-align:right;"|כאשר זכרו אנשי חכמת השיעור הוא שתהיה מרבע את קוטר הכדור ותכפול המרובע הזה ג' פעמ' ושביעית פעם ויעלה בידך {{#annot:term|814,2224|hb4l}}משיחת שטח הכדור{{#annotend:hb4l}} | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | :Multiply it by a sixth of the diameter; you receive the volume of the sphere. |
| + | :<math>\scriptstyle{\color{OliveGreen}{\rm{Surface_{sphere}}\sdot\left[\frac{1}{6}\sdot\left(2r\right)\right]}}</math> | ||
| + | |style="text-align:right;"|מנה אותו בשתות הקוטר ויעלה בידך תשבורת גוף הכדור | ||
|- | |- | ||
| | | | ||
| − | *{{#annot: | + | *{{#annot:ball|681|ol68}}Example: a sphere whose diameter is 7 cubits |
| − | |style="text-align:right;"|כגון | + | |style="text-align:right;"|כגון כדור אשר קוטרו ז' אמות{{#annotend:ol68}} |
|- | |- | ||
| | | | ||
| − | : | + | |[[File:כדור.png|thumb|150px]] |
| − | | | ||
|- | |- | ||
| − | |||
| | | | ||
| + | :The square of the diameter is 49 cubits. | ||
| + | |style="text-align:right;"|יהיה מרובע הקטר מ"ט אמה | ||
|- | |- | ||
| | | | ||
| − | : | + | :When you multiply this number 3 times and a seventh, it is 154 and this is the surface of the sphere. |
| − | : | + | :<math>\scriptstyle{\color{blue}{7^2\sdot\left(3+\frac{1}{7}\right)=49\sdot\left(3+\frac{1}{7}\right)=154}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכשאתה כופל המספר הזה ג' פעמ' ושביעית פעם יהיה קנ"ד והם מניין שטח אמות הכדור |
| − | |||
|- | |- | ||
| | | | ||
| − | :: | + | :Multiply it by a sixth of the diameter; it is 180 minus a third and this is the volume of the sphere. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{154\sdot\left(1+\frac{1}{6}\right)=180-\frac{1}{3}}}</math> |
| + | |style="text-align:right;"|מנה אותו בשתות הקוטר והוא אחד ושתות ויהיה ק"פ פחות שליש והוא תשבורת גוף הכדור הזה | ||
|- | |- | ||
| − | |< | + | | |
| − | | | + | |
| + | ==== <span style=color:green>Segment of a Sphere</span> ==== | ||
| + | |||
| + | | | ||
|- | |- | ||
| − | |||
| | | | ||
| + | :From this calculation you can deduce the volume of the segments of a sphere. | ||
| + | |style="text-align:right;"|ומתוך החשבון הזה אתה יכול להבין תשבורת שברי הכדור | ||
|- | |- | ||
| | | | ||
| − | *{{#annot: | + | *{{#annot:pool|681|T7Xc}}Example: a water pool - its body is rounded from the inside, its upper surface is a circular, its breadth [= diameter] is 7 cubits, and its depth is 3 cubits and a half. |
| − | + | |style="text-align:right;"|כגון בריכת מים אשר גופה מבפנים מעוגל ופיה עגול ורחבו ז' אמות ועומקו ג' אמות וחצי{{#annotend:T7Xc}} | |
| − | |style="text-align:right;"| | ||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :You know that this pool is a hemisphere. Multiply its depth by the breadth of its upper surface, which is the diameter of the sphere, then multiply the product you received 3 times and a seventh, as you have done with the sphere; the result is 77 and this is the surface of the hemisphere. |
| − | + | :<math>\scriptstyle{\color{blue}{\left[7\sdot\left(3+\frac{1}{2}\right)\right]\sdot\left(3+\frac{1}{7}\right)=77}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואתה יודע כי הבריכה הזאת היא חצי כדור ובא ומנה עומקה ברחב פיה אשר הוא קוטר הכדור והנקבץ בידך כפליהו ג' פעמי' ושביעית פעם כאשר עשית בכדור ויעלה ע"ז והוא {{#annot:term|2219,814|F8HS}}משיחת שטח הבריכה{{#annotend:F8HS}} |
|- | |- | ||
| | | | ||
| − | :: | + | :Multiply it by a sixth of the diameter; it is 90 minus a sixth and it is the volume of the hemispherical pool. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{77\sdot\left[\frac{1}{6}\sdot\left(2r\right)\right]=90-\frac{1}{6}}}</math> |
| + | |style="text-align:right;"|מנה אותו בשתות הקוטר יהיה צ' פחות שתות והוא תשבורת גוף הבריכה אשר היא חצי כדור | ||
|- | |- | ||
| − | |||
| | | | ||
| + | :If the depth is less than half the breadth [= less than half the diameter], know that the pool is less than a hemisphere. | ||
| + | |style="text-align:right;"|ואלו היה העומק פחות ממחצית הרוחב תהיה יודע שהבריכה הזאת מעוטה מחצי הכדור | ||
|- | |- | ||
| | | | ||
| − | + | *{{#annot:spherical solid|681|BhwX}}Example: a spherical solid [spherical cap] – its depth is 2 cubits and the breadth of the circle [= the diameter] of its base is a root of 40 which is approximately 6 and a third. | |
| − | + | |style="text-align:right;"|כגון עגולת גוף שעמקה ב' אמות ורוחב עגולת פיה גדר מ' שהוא ו' ושליש בקירוב{{#annotend:BhwX}} | |
| − | |style="text-align:right;"| | ||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :You know that this pool is less than a hemisphere, because its depth is less than half the breadth of its upper surface. |
| − | + | |style="text-align:right;"|ואתה יודע שהבריכה הזאת אין בה חצי כדור מפני שעומקה פחות ממחצית רוחב פיה | |
| − | |style="text-align:right;"| | ||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | :: | + | :If you extract its diameter as you have learned, you find it 7. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{2r=7}}</math> |
| + | |style="text-align:right;"|ואם אתה מוציא את קוטרה כאשר למדת תמצאנו ז‫' | ||
|- | |- | ||
| | | | ||
| − | + | :Multiply 2, which is the depth of the pool, by 7, which is the diameter of the sphere; it is 14. | |
| − | + | |style="text-align:right;"|ובא ומנה ב' שהוא עומק הבריכה בז' שהוא קוטר הכדור יהיו י"ד | |
| − | |style="text-align:right;"| | ||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :Multiply it 3 times and a seventh; it is 44 and this is the surface of the pool. |
| − | + | :<math>\scriptstyle{\color{blue}{\left(7\sdot2\right)\sdot\left(3+\frac{1}{7}\right)=14\sdot\left(3+\frac{1}{7}\right)=44}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כפול אותם ג' פעמי' ושביעית יהיו מ"ד והוא משיחת שטח הבריכה |
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | :: | + | :Multiply it by a sixth of the diameter, which is one and a sixth; it is 51 and a third and this is the volume of the pool. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{44\sdot\left[\frac{1}{6}\sdot\left(2r\right)\right]=44\sdot\left(1+\frac{1}{6}\right)=51+\frac{1}{3}}}</math> |
| + | |style="text-align:right;"|מנה אותו בשתות הקוטר והוא אחד ושתות יהיו נ"א ושליש והוא {{#annot:term|2219,1244|RKIL}}תשבורת גוף ה{{#annotend:RKIL}}בריכה | ||
|- | |- | ||
| − | |||
| | | | ||
| + | *{{#annot:pool|681|lEHT}}If you say that the depth of the pool is 5 cubits and the breadth [= diameter] of its upper surface is a root of 40. | ||
| + | |style="text-align:right;"|ואם היית אומר עומק הבריכה ה' אמות ורוחב פיה גדר מ‫'{{#annotend:lEHT}} | ||
|- | |- | ||
| | | | ||
| − | :{{ | + | :You find its diameter is 7. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{2r=7}}</math> |
| + | |style="text-align:right;"|היות מוצא קוטרה ז‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | :If you multiply the depth by the diameter, it is 35. |
| − | + | |style="text-align:right;"|ואם אתה מונה העומק בקוטר יהיה ל"ה | |
| − | |style="text-align:right;"| | ||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :Multiply it 3 times and a seventh; it is 110 and this is the surface of the pool. |
| − | + | :<math>\scriptstyle{\color{blue}{\left(7\sdot5\right)\sdot\left(3+\frac{1}{7}\right)=35\sdot\left(3+\frac{1}{7}\right)=110}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כפליהו ג' פעמ' ושביעית יהיה ק"י והוא משיחת שטח הבריכה |
|- | |- | ||
| | | | ||
| − | :: | + | :Multiply it by a sixth of the diameter; it is 128 and a third and this is the volume of the pool. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{110\sdot\left[\frac{1}{6}\sdot\left(2r\right)\right]=128+\frac{1}{3}}}</math> |
| + | |style="text-align:right;"|מנה אותו בשתות הקוטר יהיה קכ"ח ושליש והוא {{#annot:term|2219,1244|SpiW}}תשבורת רבוע ה{{#annotend:SpiW}}בריכה וכה"ל | ||
|- | |- | ||
| − | | | + | | |
| + | ==== <span style=color:green>Triangular Prism</span> ==== | ||
| + | |||
| | | | ||
|- | |- | ||
| | | | ||
| − | : | + | :97) A halved prism: a triangular prism is called halved, bacause it is half a square prism. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|צז {{#annot:triangular|1100,2213|OosO}}<big>מצבה</big> חצויה{{#annotend:OosO}} וקורים ל{{#annot:triangular|1100,2213|rw6P}}מצבה משולשת חצויה{{#annotend:rw6P}} מפני שהיא מחצית המרובעת |
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | *{{#annot:prism|681|Gs8u}}A halved prism, its upper base and its lower base are a right triangle, whose one side is 3 cubits, the second [side] is 4 [cubits] and the third [side] is 5 [cubits], and the height [of the prism] is 10 cubits |
| − | | | + | |style="text-align:right;"|מצבה חצויה אשר ראשה ותושבתה {{#annot:triangle|1104,1341|YJQE}}משולש נצב הזוית{{#annotend:YJQE}} אשר צלעו האחת ג' אמות והשני ד' והשלישית ה' וגובהו י' אמות{{#annotend:Gs8u}} |
| − | | | ||
| − | |||
|- | |- | ||
| | | | ||
| − | + | :[[File:Kelalim ba-Mispar 97-1.png|thumb|70px|left]]<br> | |
| − | + | <span style=color:red>[this figure does not appear in the text]</span> | |
| + | | | ||
|- | |- | ||
| | | | ||
| − | : | + | :You know the area of this triangle is 6 cubits. |
| − | | | + | |style="text-align:right;"|אתה יודע {{#annot:term|816,1244|pgiu}}תשבורת{{#annotend:pgiu}} המשולש הזה והוא ו' אמות |
|- | |- | ||
| | | | ||
| − | :: | + | :Multiply it by 10, which is the height; it is 60 cubits and this is the volume of the halved prism. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{OliveGreen}{\rm{height_{prism}}\times \rm{Area_{triangle}}}}{\color{blue}{=10\sdot6=60}}</math> |
| + | |style="text-align:right;"|מנה אותם בי' שהוא הגובה יהיו ס' אמה והוא תשבורת ה{{#annot:triangular|1100,2213|FrRB}}מצבה חצוית הראש{{#annotend:FrRB}} | ||
|- | |- | ||
| | | | ||
| − | + | ||
| − | + | ==== <span style=color:green>Pentagonal / Hexagonal Prism</span> ==== | |
| − | + | ||
| − | |||
| | | | ||
|- | |- | ||
| | | | ||
| − | : | + | :Likewise if the upper and lower bases of the solid are pentagonal or hexagonal surfaces, it is called pentagonal or hexagonal prism. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכן אלו היה ה{{#annot:term|587,2151|5Z9l}}גולם{{#annotend:5Z9l}} ראשו ותושבתו שטחים מחומשות או משושתות היא נקראת {{#annot:pentagonal|1100,1672|K9aE}}מצבה מחומשת{{#annotend:K9aE}} או משותתת |
|- | |- | ||
| | | | ||
| − | : | + | :You know the area of the pentagon or the hexagon that is its lower or upper base. You multiply its area by the height and you receive the volume of that prism. |
| − | | | + | |style="text-align:right;"|תהיה יודע {{#annot:term|816,2224|DJo0}}משיחת ה{{#annotend:DJo0}}מחומש או המשותת הזה אשר היא תושבתו או ראשו ותהיה מונה משיחתו במניין הגובה ויעלה בידך תשבורת ה{{#annot:term|1100,2212|NojB}}מצבה{{#annotend:NojB}} ההיא |
|- | |- | ||
| − | | | + | | colspan="2"| |
| − | + | :<math>\scriptstyle{\color{OliveGreen}{\rm{Volume_{prism}=height_{prism}}\times\rm{Area_{pentagon/hexagon}}}}</math> | |
|- | |- | ||
| | | | ||
| − | + | ||
| − | + | ==== <span style=color:green>Cylinder</span> ==== | |
| + | |||
| + | | | ||
|- | |- | ||
| | | | ||
| − | :: | + | :If the upper and lower bases of the solid are circular surfaces and the whole solid rises circularily to the top: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואם יהיה הגולם ראשו ותשברתו שטחים עגולים וכל גופו עולה בעגול עם הראש |
|- | |- | ||
| − | |||
| | | | ||
| + | :You calculate the volume of this shape the way you find the previous shapes. | ||
| + | |style="text-align:right;"|ואתה מונה תשבורת התמונה הזאת על הדרך אשר ידעת התמונות הראשונות | ||
|- | |- | ||
| | | | ||
| − | :{{ | + | :If you find the area of the circle that is the lower or upper base, then multiply its area by the height, you get the volume of this shape called a cylinder [lit. circular prism]. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{OliveGreen}{\rm{Volume_{cylinder}=height_{cylinder}}\times\rm{Area_{circle}}}}</math> |
| + | |style="text-align:right;"|אם אתה מרבע העגול שהוא התושבת או הראש ואתה מונה מרובעו במניין הגובה יעלה בידך תשבורת התמונה ‫<ref>98v</ref>הזאת והיא נקראת {{#annot:term|1101,1471|zGsp}}מצבה עגולה{{#annotend:zGsp}} | ||
|- | |- | ||
| − | |the | + | | |
| − | |style="text-align:right;"| | + | *{{#annot:cylinder|681|nprV}}Example: a cylinder, its upper base as well as its lower base are a circle whose diameter is 10 cubits, and the height of the cylinder is 14. |
| + | |style="text-align:right;"|ואני נותן לך דמיון מזה {{#annot:term|1101,1471|Mt2g}}מצבה עגולה {{#annotend:Mt2g}}אשר ראשה וכן תושבתה שטח עגול אשר קוטרו י' אמות וגובה המצבה י"ד{{#annotend:nprV}} | ||
|- | |- | ||
| | | | ||
| − | + | :[[File:Kelalim ba-Mispar 97-2.png|thumb|70px|left]] | |
| − | |style="text-align:right;"| | + | :<span style=color:red>[this figure does not appear in the text]</span> |
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :You can get the volume of this prism, if you multiply the diameter of the circle, which is 10 cubits, by itself; it is 100 cubits. You multiply 100 by 14 that are the cubits of the height; it is 1400 cubits and this would be the volume of this shape if it were square. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\left(2r\right)^2\sdot\rm{height_{cylinder}}}}{\color{blue}{=10^2\sdot14=100\sdot14=1400}}</math> | ||
| + | |style="text-align:right;"|ואתה יכול להגיע אל תשבורת המצבה הזאת אם אתה מונה את קוטר העגול שהוא י' אמות במנין עצמם ויהיו ק' אמות ומנה ק' בי"ד שהם אמות הגובה ויהיו אלף ות' אמות וזה הוא תשבורת התמונה הזאת אלו היתה מרובעת | ||
|- | |- | ||
| | | | ||
| − | : | + | :Subtract its seventh and its half-seventh from this number, which is 300 cubits; you are left with 110 cubits and that is the volume of the cylinder. |
| − | |[[ | + | |style="text-align:right;"|הוצא מהמספר הזה שבעיתו וחצי שבעיתו והם ג' מאות אמה ונשאר בידך אלף ומאה אמה והוא תשבורת המצבה העגולה |
| + | |- | ||
| + | | colspan="2"| | ||
| + | :<math>\scriptstyle{\color{blue}{1400-\left[\left[\frac{1}{7}+\left(\frac{1}{2}\sdot\frac{1}{7}\right)\right]\sdot1400\right]=1400-300=1100}}</math> | ||
|- | |- | ||
| | | | ||
| − | : | + | :Also if you were to subtract from 100 cubits, which is a quarter of the base, its seventh and its half-seventh, which is 2[1] cubits and 3 parts of 7 parts of a cubits, you would have been left with 78 cubits and [4] parts of 7 of a cubits and this is the volume of the circle that is the base of the prism. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכן אלו היית מוציא מן ק' אמה שהוא רביע התושבת שביעיתו ומחצית שבעיתו והוא '''כ'''' אמה וג' חלקי' מז' חלקי' באמה וישאר בידך ע"ח אמה ו'''ג'''' חלקי' מז' באמה והם {{#annot:term|816,2224|INwY}}משיחת ה{{#annotend:INwY}}עגול אשר היא תושבת המצבה |
| + | |- | ||
| + | | colspan="2"| | ||
| + | :<math>\scriptstyle{\color{blue}{100-\left[\left[\frac{1}{7}+\left(\frac{1}{2}\sdot\frac{1}{7}\right)\right]\sdot100\right]=100-\left(21+\frac{3}{7}\right)=78+\frac{4}{7}}}</math> | ||
|- | |- | ||
| − | |||
| | | | ||
| + | :Multiply it by the height, which is 14; the result is 110 cubits and it is the volume of the cylinder. | ||
| + | |style="text-align:right;"|מנה אותה בגובה אשר הוא י"ד יעלה המניין לאלף ומאה אמה והוא תשבורת המצבה הזאת העגולה | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\rm{Volume_{circle}}\times \rm{height_{cylinder}}}}{\color{blue}{=\left(78+\frac{4}{7}\right)\sdot14=1100}}</math> | ||
|- | |- | ||
| | | | ||
| − | :{{ | + | :<span style=color:green>General rule:</span> You see that the practice with the whole species is the same: if you know the area of the base of any shape, whether the upper or the lower base, and you multiply its area by the height, you find the volume of that solid. There is no difference there. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{OliveGreen}{\rm{Volume_{prism}=Area_{base}}\times\rm{height_{prism}}}}</math> |
| − | + | |style="text-align:right;"|ונראה לך שדרך כל המין הזה נוהג מנהג אחד ואם אתה יודע מספר תשבורת מזה התושבת מן התושבת או הראש מאיזו תמונה הייתה ותמנה תשברתה במנין הגובה י' אתה מוצא תשבורת ה{{#annot:term|587,1445|EyrJ}}גוף{{#annotend:EyrJ}} ההוא ואין שם חילוף | |
|- | |- | ||
| + | ! | ||
| + | |||
| + | === Questions about Rectangles === | ||
| + | |||
| + | |style="text-align:right;"|<big>שאלות</big> במרובע ארוך | ||
| + | |- | ||
| + | !<span style=color:green>Figure Problem - Diagonal - Rectangle</span> | ||
| | | | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :{{#annot:rectangle|683|407S}}98) A rectangle whose one side is 8 cubits and its other side is 6 [cubits]. |
| − | |style="text-align:right;"| | + | :How much is its diagonal? |
| + | |style="text-align:right;"|צח {{#annot:term|591,1525|KIEb}}מרובע ארוך{{#annotend:KIEb}} שיש בצלעו האחד ח' אמות ובצלעו השנית ו‫'<br> | ||
| + | כמה הוא אלכסונו{{#annotend:407S}} | ||
|- | |- | ||
| | | | ||
| − | : | + | :[[File:Kelalim ba-Mispar 98.png|thumb|150px|left]] |
| − | | | + | |[[File:כללים במספר - צח.png|thumb|150px]] |
|- | |- | ||
| | | | ||
| − | : | + | :The answer: multiply 8, which is one side, by 6, which is the other side; their product is 48. |
| − | | | + | |style="text-align:right;"|תשובה מנה ח' שהוא צלע האחת בו' שהוא הצלע השנית ויהיה רבועם מ"ח |
|- | |- | ||
| | | | ||
| − | : | + | :Double this product; it is 96. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכפול המרובע הזה והם צ"ו |
|- | |- | ||
| − | |||
| | | | ||
| + | :If you add to it the square of 2, which is the excess of one side over the other, the total is 100. | ||
| + | |style="text-align:right;"|ואם {{#annot:term|178,1206|xJzM}}תוסיף עליו{{#annotend:xJzM}} מרובע ב' אשר היא {{#annot:term|877,1207|Vj4x}}תוספת{{#annotend:Vj4x}} הצלע האחת אל השנית יהיה הכל ק‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | :The root of 100 is 10 and it is the diagonal of this quadrilateral. |
| − | + | |style="text-align:right;"|וגדר ק' הוא י' והוא {{#annot:term|1106,1878|VGc4}}אלכסון{{#annotend:VGc4}} מן המרובע הזה | |
| − | |style="text-align:right;"| | ||
| − | |||
|- | |- | ||
| − | | | + | |colspan=2| |
| − | + | :<math>\scriptstyle{\color{blue}{\sqrt{\left[2\sdot\left(8\sdot6\right)\right]+\left(8-6\right)^2}=\sqrt{\left(2\sdot48\right)+2^2}=\sqrt{96+2^2}=\sqrt{100}=10}}</math> | |
|- | |- | ||
| | | | ||
| − | + | :The general rule of this matter is that for every rectangle, if you add the square of the difference between the two sides to twice its area, the sum both is equal to the square of its diagonal. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכללו של ענין שכל מרובע ארוך אם אתה מוסיף רבוע העודף אשר בן שתי צלעיו על כפל תשברתו יהיו אלה שניהם שוים למרובע אלכסונו |
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\rm{diagonal_{rectangle}}=\sqrt{\left(2\sdot\rm{Area_{rectangle}}\right)+\left(\rm{side_1-side_2}\right)^2}}}</math> | ||
|- | |- | ||
| + | !<span style=color:green>Figure Problem - Side; Area - Rectangle</span> | ||
| | | | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :{{#annot:rectangle|680,679|noAQ}}99) Another question: a rectangle whose diagonal is 10 cubits, and its length exceeds its breadth by 2 cubits. |
| − | |style="text-align:right;"| | + | :How much are its length, its breadth, and its area? |
| + | |style="text-align:right;"|צט <big>שאלה</big> אחרת מרובע ארוך אשר באלכסונו י' אמה וארכו מוסיף על רחבו ב' אמות<br> | ||
| + | כמה הוא ארכו וכמה הוא רחבו וכמה תשברתו{{#annotend:noAQ}} | ||
|- | |- | ||
| − | |||
| | | | ||
| + | :[[File:Kelalim ba-Mispar 99.png|thumb|150px|left]] | ||
| + | |[[File:כללים במספר - צט.png|thumb|200px]] | ||
|- | |- | ||
| | | | ||
| − | : | + | :Answer: you know that the square of the diagonal is 100. |
| − | + | |style="text-align:right;"|תשובה אתה יודע כי מרובע האלכסון הוא ק‫' | |
| − | |||
| − | |style="text-align:right;"| | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :Subtract from it the square of the excess of the length over the breadth, which is 2 and its square is 4; you are left with 96. |
| − | | | + | |style="text-align:right;"|הוצא ממנו רבוע עדף האורך על הרחב אשר הוא ב' וריבועו ד' ישאר בידך מהם צ"ו |
|- | |- | ||
| − | |the area of the | + | | |
| − | |style="text-align:right;"| | + | :Divide it in half; it is 48 and this is the area of the quadrilateral. |
| + | |style="text-align:right;"|חלק אותם לשנים ויהיו מ"ח והוא תשבורת המרובע | ||
|- | |- | ||
| − | | | + | |colspan=2| |
| − | : | + | :<math>\scriptstyle{\color{blue}{\frac{1}{2}\sdot\left[diagonal^2-\left(side_1-side_2\right)^2\right]=\frac{1}{2}\sdot\left(10^2-2^2\right)=\frac{1}{2}\sdot\left(100-4\right)=\frac{1}{2}\sdot96=48}}</math> |
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :If you wish to know its sides, one of which exceeds over the other by 2, divide this excess in half; it is 1 and its square is 1. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואם תרצה לדעת צלעותיו אשר האחד מוספת על השנית ב' בא וחלק העודף הזה לשנים ויהיה א' וריבועו א‫' |
|- | |- | ||
| − | |||
| | | | ||
| + | :Add it to the area; it is 49. | ||
| + | |style="text-align:right;"|הוסיפנו על התשבורת ויהיה מ"ט | ||
|- | |- | ||
| | | | ||
| − | : | + | :The root of this number is 7. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וגדר המניין הזה הוא ז‫' |
|- | |- | ||
| | | | ||
| − | : | + | :If you add to it one, which is half the excess, it is 8 and this is the length. |
| − | | | + | |style="text-align:right;"|ואם תוסיף עליו אחת שהוא מחצית העודף יהיה ח' והוא קו האורך |
|- | |- | ||
| − | | | + | |colspan=2| |
| − | <math>\scriptstyle\left(\frac{1}{ | + | :<math>\scriptstyle{\color{blue}{\sqrt{\left(\frac{1}{2}\sdot2\right)^2+\rm{Area}}+\left(\frac{1}{2}\sdot2\right)=\sqrt{1^2+48}+1=\sqrt{1+48}+1=\sqrt{49}+1=7+1=8}}</math> |
| − | |||
|- | |- | ||
| | | | ||
| − | + | :If you subtract 1 from it, 6 remains and it is the breadth. | |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{\sqrt{\left(\frac{1}{2}\sdot2\right)^2+\rm{Area}}-\left(\frac{1}{2}\sdot2\right)=7-1=6}}</math> |
| + | |style="text-align:right;"|ואם תפחות ממנו א' ישאר ו' והוא קו הרוחב | ||
|- | |- | ||
| | | | ||
| − | : | + | :The product of 8 by 6 is 48 and this is the area. |
| − | + | :<math>\scriptstyle{\color{blue}{8\sdot6=48}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ומנין ח' בו' הוא מ"ח והוא התשבורת |
| − | |||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | | |
| − | + | *{{#annot:rectangle|679|GcE4}}If one says: A rectangle, its area is 48 and the sum of its length and its breadth together is 14. | |
| − | |style="text-align:right;"|ואם | + | :How much are its length and its breadth? |
| + | |style="text-align:right;"|ואם יאמר מרובע בתשברתו מ"ח וקו ארכו עם קו רחבו שניהם יחד י"ד<br> | ||
| + | כמה הוא ארכו וכמה הוא רחבו{{#annotend:GcE4}} | ||
|- | |- | ||
| | | | ||
| − | + | :To answer this question, take a half of 14, it is 7. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ולתשובת השאלה הזאת תקח מחצית י"ד והוא ז‫' |
|- | |- | ||
| | | | ||
| − | : | + | :Square it; it is 49. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ותרבע אותו ויהיה מ"ט |
|- | |- | ||
| | | | ||
| − | : | + | :Subtract from it 48, which is the area; you are left with 1. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|הוצא מהם מ"ח הוא התשבורת וישאר בידך א‫' |
|- | |- | ||
| − | |||
| | | | ||
| + | :The root of 1 it 1. | ||
| + | |style="text-align:right;"|וגדר הא' הוא א‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | :If you add it to 7, it is 8 and this is the length of the quadrilateral. |
| − | + | |style="text-align:right;"|אם תוסיפנו על ז' יהיה ח' והוא אורך המרובע | |
| − | |style="text-align:right;"| | + | |- |
| − | + | |colspan=2| | |
| + | :<math>\scriptstyle{\color{blue}{\left(\frac{1}{2}\sdot14\right)+\sqrt{\left(\frac{1}{2}\sdot14\right)^2-48}=7+\sqrt{7^2-48}=7+\sqrt{49-48}=7+\sqrt{1}=7+1=8}}</math> | ||
|- | |- | ||
| | | | ||
| − | : | + | :If you subtract one from 7, you are left with 6 and this is the breadth of the quadrilateral. |
| − | | | + | :<math>\scriptstyle{\color{blue}{\left(\frac{1}{2}\sdot14\right)-\sqrt{\left(\frac{1}{2}\sdot14\right)^2-48}=7-1=6}}</math> |
| + | |style="text-align:right;"|ואם תפחות האחד מן הז' ישאר בידך ו' והוא רוחב המרובע | ||
|- | |- | ||
| | | | ||
| − | : | + | :Know that if a question is asked on this matter, in which the area exceeds the square of half [the sum of] the sides, this question is false. |
| − | + | |style="text-align:right;"|והוי יודע כי אם ישאל בענין זה שאלה אשר יהיה בה התשבורת עודף על מרובע מחצית הצלעות כי שאלה זאת שקר וכזב | |
| − | |style="text-align:right;"| | ||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| − | |the | + | | |
| − | |style="text-align:right;"| | + | *{{#annot:rectangle|679|IPeT}}Example: one says to you: a rectangle whose area is 48 and the sum of its length and its breadth together is 13. |
| + | |style="text-align:right;"|כגון האומר לך מרובע שתשברתו מ"ח וקו ארכו עם רחבו י"ג{{#annotend:IPeT}} | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | :He is wrong, or testing you, and you do not have to answer. |
| + | |style="text-align:right;"|טעות הוא בידו ‫<ref>99r</ref>או מנסה הוא לך ואינך צריך להשיב | ||
|- | |- | ||
| − | |< | + | | |
| − | | | + | |
| + | === <span style=color:green>Square</span> === | ||
| + | |||
| + | | | ||
|- | |- | ||
| − | + | !<span style=color:green>Figure Problem - Diagonal - Square</span> | |
| | | | ||
|- | |- | ||
| | | | ||
| − | :{{#annot: | + | :{{#annot:square|683|rMZ5}}100) Question: a square, its length is 10 and its breadth is 10. |
| − | |style="text-align:right;"| | + | :How much is its diagonal? |
| − | + | |style="text-align:right;"|ק <big>שאלה</big> מרובע השוה ונצב הצ' הזוית שארכו י' ורחבו י‫'<br> | |
| − | + | כמה הוא אורך אלכסונו{{#annotend:rMZ5}} | |
| − | |||
|- | |- | ||
| | | | ||
| − | + | :Answer: the diagonal is a root of two hundred, because the square of the diagonal is two hundred. | |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{\sqrt{2\sdot10^2}=\sqrt{200}}}</math> |
| − | + | |style="text-align:right;"|תשובה האלכסון הזה הוא גדר מאתים כי מרובע האלכסון ימצא ברבועו מאתי‫' | |
| − | |||
|- | |- | ||
| | | | ||
| − | :: | + | :Since, for every square [lit. right-angled quadrilateral], the square of its diagonal is twice that square. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{OliveGreen}{\left(\rm{diagonal_{\square}}\right)^2=2\sdot\left(\rm{side_{\square}}\right)^2}}</math> |
| + | |style="text-align:right;"|מפני שכל מרובע נצב הזוויות רבוע אלכסונו כפלים מהמרובע ההוא | ||
|- | |- | ||
| | | | ||
| − | : | + | :So, we say about this diagonal that it is the root of two hundred, which is twice the square of 10 by 10. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ומפני זה אמרנו על האלכסון הזה שהוא גדר מאתיי' אשר הוא כפלים מרובע י' על י‫' |
|- | |- | ||
| + | !<span style=color:green>Figure Problem - Side - Square</span> | ||
| | | | ||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | | |
| − | + | *{{#annot:square|679|LjXT}}If it is said: A square whose diagonal is √200. | |
| − | |style="text-align:right;"| | + | :How much is its side? |
| + | |style="text-align:right;"|ואם יאמר מרובע שאלכסונו גדר מאתים<br> | ||
| + | כמה צלעו{{#annotend:LjXT}} | ||
|- | |- | ||
| | | | ||
| − | : | + | :Divide the square of the diagonal in half; it is 100. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|חלק מרובע האלכסון לשנים ויהיה ק' האחד |
|- | |- | ||
| | | | ||
| − | ::the side = | + | :Say: the root of 100 is the side of the square and it is 10. |
| − | + | |style="text-align:right;"|ואמור גדר ק' הוא הצלע המרובע והוא י‫' | |
| − | + | |- | |
| − | + | |colspan=2| | |
| − | + | :<math>\scriptstyle{\color{blue}{\sqrt{\frac{1}{2}\sdot\rm{diagonal}^2}=\sqrt{\frac{1}{2}\sdot200}=\sqrt{100}=10}}</math> | |
|- | |- | ||
| − | |||
| | | | ||
| + | :This diagonal is 14 and a seventh approximately. | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{200}\approx14+\frac{1}{7}}}</math> | ||
| + | |style="text-align:right;"|והאלכסון הזה י"ד ושביעית בקרוב | ||
|- | |- | ||
| + | !<span style=color:green>Figure Problem - Side; Area - Square</span> | ||
| | | | ||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | ::the | + | :{{#annot:square|680,679|VUWx}}101) Another question: a square, you subtract the sum of its four sides from its area and the remainder is 21 cubits. |
| − | : | + | :How much is its area, and how much is each of its sides? |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|קא <big>שאלה</big> אחרת מרובע שוה שהוצאת מן מניין תשברתו מניין צלעותיו הארבעה ונשאר בידך מהתשבורת כ"א אמה<br> |
| − | + | כמה הוא התשבורת וכמה מניין כל צלע וצלע מהמרובע{{#annotend:VUWx}} | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| − | |||
| | | | ||
| + | :Answer: divide the number of the sides, which is 4, in half. | ||
| + | |style="text-align:right;"|תשובה חלק מנין הצלעות שהוא ד' לשנים | ||
|- | |- | ||
| | | | ||
| − | + | :Multiply two by itself, meaning square it; it is 4. | |
| − | + | |style="text-align:right;"|ומנה השנים בעצמם כלומר רבעם יהיו ד‫' | |
| − | |style="text-align:right;"| | ||
| − | |||
|- | |- | ||
| − | |the | + | | |
| − | |style="text-align:right;"| | + | :Add to this number the number that is given to you that remains from the square; the total is 25. |
| + | |style="text-align:right;"|הוסף המניין הזה על המניין המסודר לך הנשאר לך מן המרובע ויהיה הכל כ"ה | ||
|- | |- | ||
| | | | ||
| − | : | + | :Find the root of 25; it is 5. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ודע גדר כ"ה והוא ה‫' |
| − | |||
|- | |- | ||
| − | |||
| | | | ||
| + | :Add to it half the number of the sides, which is 2; the total is 7 and it is the side of the square. | ||
| + | |style="text-align:right;"|ותוסיף עליו חצי מניין הצלעות והוא ב' ויהיה הכל ז' והוא צלע המרובע | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{\left(\frac{1}{2}\sdot4\right)^2+21}+\left(\frac{1}{2}\sdot4\right)=\sqrt{2^2+21}+2=\sqrt{4+21}+2=\sqrt{25}+2=5+2=7}}</math> | ||
|- | |- | ||
| | | | ||
| − | :{{ | + | :Its area is 49. |
| − | + | :<math>\scriptstyle{\color{blue}{7^2=49}}</math> | |
| − | + | |style="text-align:right;"|ותשברתו מ"ט | |
| − | |style="text-align:right;"| | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | *{{#annot:square|680|4RxR}}If it is said: A square, you add the sum of its four sides to its area and the result is 77. |
| − | + | :How much is the area? | |
| + | |style="text-align:right;"|ואם יאמר מרובע אשר הוספת מניין כל ד' צלעותיו על מניין תשברתו ויהיה הכל ע"ז<br> | ||
| + | כמה הוא המרובע הזה{{#annotend:4RxR}} | ||
|- | |- | ||
| | | | ||
| − | : | + | :In this question, take half the number of the sides; it is 2. |
| − | + | |style="text-align:right;"|ואתה בשאלה הזאת קח מניין חצי הצלעות והוא ב‫' | |
| − | |style="text-align:right;"| | ||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :Multiply it by itself; it is 4. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ותמנה אותו בעצמו ויהיו ד‫' |
|- | |- | ||
| | | | ||
| − | : | + | :Add it to the number that is given to you, which is 77; it is 81. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והוסיפנו על המניין שמסר לך והוא ע"ז ויהיו פ"א |
|- | |- | ||
| | | | ||
| − | : | + | :Take the root of this number; it is 9. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וקח גדר המספר הזה והוא ט‫' |
|- | |- | ||
| | | | ||
| − | + | :Subtract from it half the number of the sides that you added; you are left with 7 and it is the side of the square. | |
| − | + | |style="text-align:right;"|הוצא ממנו חצי מספר הצלעות שהוספת וישארו בידך ז' והוא צלע המרובע | |
| − | + | |- | |
| − | |style="text-align:right;"| | + | |colspan=2| |
| − | + | :<math>\scriptstyle{\color{blue}{\sqrt{\left(\frac{1}{2}\sdot4\right)^2+77}-\left(\frac{1}{2}\sdot4\right)=\sqrt{2^2+77}-2=\sqrt{4+77}-2=\sqrt{81}-2=9-2=7}}</math> | |
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :Its area is 49. |
| − | + | :<math>\scriptstyle{\color{blue}{7^2=49}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ותשברתו מ"ט |
| − | |||
| − | |||
|- | |- | ||
| − | + | !<span style=color:green>Figure Problem - Side - Square</span> | |
| | | | ||
|- | |- | ||
| | | | ||
| − | :{{#annot: | + | :{{#annot:square|679|CMcq}}102) The third question: a square, you subtract its area from the sum of its four sides and the remainder is 3. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle4a-a^2=3</math> |
| + | |style="text-align:right;"|קב <big>שאלה</big> שלישית מרובע השלכת תשברתו ממניין ד' צלעותיו ונשאר בידך ג‫'{{#annotend:CMcq}} | ||
|- | |- | ||
| | | | ||
| − | : | + | :Divide the number of the sides by 2; their half is 2. |
| − | | | + | |style="text-align:right;"|חלק מניין הצלעות על ב' וחציים הוא ב‫' |
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | :Its square is 4. |
| + | |style="text-align:right;"|וריבועם הוא ד‫' | ||
|- | |- | ||
| − | |||
| | | | ||
| + | :Subtract from it the three you have left; 1 remains. | ||
| + | |style="text-align:right;"|הוצא מהם השלשה שנשארו בידך וישאר א‫' | ||
|- | |- | ||
| − | | | + | | |
| − | + | :Its root is 1. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אשר גדרו א‫' |
| − | |||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | :Subtract it from half [the number of] the sides; one remains and this is the side of the square. |
| − | + | |style="text-align:right;"|פחות אותו ממחצית הצלעות וישאר אחד והוא צלע המרובע | |
|- | |- | ||
| − | | | + | |colspan=2| |
| − | + | :<math>\scriptstyle{\color{blue}{\left(\frac{1}{2}\sdot4\right)-\sqrt{\left(\frac{1}{2}\sdot4\right)^2-3}=2-\sqrt{2^2-3}=2-\sqrt{4-3}=2-\sqrt{1}=2-1=1}}</math> | |
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | :Or, add the root of the one you have left to half [the number of] the sides; it is three and this is also the side of the square. |
| + | :<math>\scriptstyle{\color{blue}{\left(\frac{1}{2}\sdot4\right)+\sqrt{\left(\frac{1}{2}\sdot4\right)^2-3}=2+\sqrt{1}=2+1=3}}</math> | ||
| + | |style="text-align:right;"|או הוסף עליו גדר האחד שנשאר בידך על מחצית הצלעות יהיו ג' ויהיו גם הם צלע המרובע | ||
|- | |- | ||
| | | | ||
| − | : | + | :Since 1 and 3 can be the root, so there are two possible answers to this question. |
| − | + | |style="text-align:right;"|כי יכול יהיה א' הגדר ויכול יהיה ג' כי שני חשבונות לשאלה הזאת | |
| − | |style="text-align:right;"| | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | + | ||
| − | + | === <span style=color:green>Area</span> === | |
| − | + | ||
| − | + | | | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | | | ||
| − | |||
|- | |- | ||
| | | | ||
| − | + | ==== <span style=color:green>Circle</span> ==== | |
| − | + | ||
| − | + | | | |
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :{{#annot:circle|680|FmNq}}103) If you want to know the area of a whole circle, when its diameter is known |
| − | + | |style="text-align:right;"|קג <big>אם</big> תרצה לדעת תשבורת ''העגול התמים'' אם תדע {{#annot:term|1107,1878|vRfG}}אלכסונו{{#annotend:vRfG}} והוא הקוטר{{#annotend:FmNq}} | |
| − | |style="text-align:right;"| | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | :: | + | :Multiply it by 3 and a seventh and this is the length of the perimeter. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{OliveGreen}{2r\sdot\left(3+\frac{1}{7}\right)}}</math> |
| + | |style="text-align:right;"|תכפול אותו ג' פעמי' ושבעית פעם והוא יהיה אורך הקו הסובב | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{ | + | :Then square half the diameter by half the perimeter and this is area of the circle. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{OliveGreen}{\left(\frac{1}{2}\sdot2r\right)\sdot\left(\frac{1}{2}\sdot \rm{perimeter_{\bigcirc}}\right)}}</math> |
| + | |style="text-align:right;"|ואחר כן הוי מרבע מחצית הקוטר במחצית הקו הסובב והוא יהיה תשבורת העגול | ||
|- | |- | ||
| | | | ||
| − | + | *{{#annot:circle|680|KGxZ}}Example: a circle whose diameter is 14. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והדמיון לענין הזה עגולה שהקוטר שלה י"ד{{#annotend:KGxZ}} |
|- | |- | ||
| − | |||
| | | | ||
| + | :[[File:Kelalim ba-Mispar 103.png|thumb|170px|left]] | ||
| + | |[[File:כללים במספר - קג.png|thumb|170px]] | ||
|- | |- | ||
| | | | ||
| − | : | + | :Multiply it by 3 and a seventh; it is 44 and this is the measure of the perimeter. |
| − | : | + | :<math>\scriptstyle{\color{blue}{14\sdot\left(3+\frac{1}{7}\right)=44}}</math> |
| − | + | |style="text-align:right;"|ואתה כופל אותו ג' פעמ' ושביעית ויהיה מ"ד והוא מדת הקו הסובב | |
| − | |||
| − | |||
| − | |||
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | :If you multiply half the diameter, which is 7, by half the perimeter, which is 22, the product is 154 cubits and this is area of the circle. | |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{\left(\frac{1}{2}\sdot14\right)\sdot\left(\frac{1}{2}\sdot44\right)=7\sdot22=154}}</math> |
| + | |style="text-align:right;"|ואם אתה מונה מחצית הקוטר והוא ז' במחצית הקו הסובב והוא כ"ב יהיה המניין קנ"ד אמות והוא תשבורת העגול | ||
|- | |- | ||
| | | | ||
| − | : | + | ==== <span style=color:green>Triangle</span> ==== |
| − | + | ||
| − | |||
| | | | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | :: | + | :{{#annot:triangle|680|akTZ}}104) You have a triangle such as this and you want to know its area. |
| − | + | |style="text-align:right;"|קד <big>הרי</big> שיש לפניך אי' משולש כזה ותרצה לידע מדת שבריו{{#annotend:akTZ}} | |
| − | |||
| − | |style="text-align:right;"| | ||
| − | |||
|- | |- | ||
| − | |||
| | | | ||
| + | :[[File:Kelalim ba-Mispar 104.png|thumb|170px|left]] | ||
| + | |[[File:כללים במספר - קד.png|thumb|170px]] | ||
|- | |- | ||
| | | | ||
| − | : | + | :Multiply half the upper side by the whole height and this is the measure of its area. |
| − | + | :<math>\scriptstyle{\color{OliveGreen}{\left(\frac{1}{2}\sdot side\right)\sdot \rm{height_{\triangle}}}}</math> | |
| − | + | |style="text-align:right;"|תרבה חצי צלע העליון על כל ה{{#annot:term|1111,2206|iYwS}}עמוד{{#annotend:iYwS}} וכן יהיו מדת שבריו | |
| − | |||
| − | |style="text-align:right;"| | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | *{{#annot:triangle|680| | + | *{{#annot:triangle|680|sIAa}}Example: the upper side is 10 cubits and its height is 12 cubits. |
| − | |style="text-align:right;"|המשל בזה | + | |style="text-align:right;"|המשל בזה הרי שהיה צלע העליון י' אמות והעמוד שלו י"ב אמות{{#annotend:sIAa}} |
|- | |- | ||
| | | | ||
| − | : | + | :Take half the upper side, which is 5 and multiply it by the whole height, which is 12, say: 12 times 12 is 60 and this is the result. |
| − | | | + | :<math>\scriptstyle{\color{blue}{\left(\frac{1}{2}\sdot10\right)\sdot12=5\sdot12=60}}</math> |
| + | |style="text-align:right;"|תקח ‫<ref>99v</ref>חצי צלע העליון שהוא ה' ותרבה אותו על כל העמוד שהוא י"ב ואמור ה' פעמ' י"ב ס' וכן יבא | ||
|- | |- | ||
| | | | ||
| − | + | ==== <span style=color:green>Square</span> ==== | |
| − | + | ||
| − | |||
| | | | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| − | |||
| | | | ||
| + | :{{#annot:square|680|Eple}}105) An equilateral quadrangle whose angles are right angles - you want to know its area | ||
| + | |style="text-align:right;"|קה <big>המרובע</big> שהוא שוה הצלעות וכל זוויותיו נצבות ותרצה לידע תשברתו{{#annotend:Eple}} | ||
|- | |- | ||
| | | | ||
| − | : | + | :Measure one of its sides and multiply it by itself and [its area is] the result. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{OliveGreen}{\rm{side}^2}}</math> |
| + | |style="text-align:right;"|תמדוד אחד מצלעיו ותרבה אותו בעצמו וכפי מה שיעלה כן יהיה | ||
|- | |- | ||
| | | | ||
| − | : | + | *{{#annot:square|680|J0ct}}Example: a square, each of its sides is 4. |
| − | + | |style="text-align:right;"|המשל בזה מרובע אחד שכל אחד מצלעותיו ד' וזוויותיו נצבות{{#annotend:J0ct}} | |
| − | |||
| − | |||
| − | |||
| − | |style="text-align:right;"| | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | :: | + | :[[File:Kelalim ba-Mispar 105.png|thumb|100px|left]] |
| − | | | + | |[[File:כללים במספר - קה.png|thumb|100px]] |
|- | |- | ||
| | | | ||
| − | ::the area | + | :Measure one of its sides, which is 4, multiply it by itself, say: 4 times 4 is 16 and this is the measure of its area. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{4^2=16}}</math> |
| + | |style="text-align:right;"|תמדוד אחד מצלעותיו שהוא ד' ותרבה אותו בעצמו ואמור ד' פעמ' ד' י"ו וכן יהיה מניין תשברתו וכה"ל | ||
|- | |- | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| | | | ||
| − | + | ==== <span style=color:green>Triangle whose base is an arc - Sector</span> ==== | |
| + | |||
| | | | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | :: | + | :{{#annot:sector|680|Eekc}}106) A triangle whose base is arc BG and its two sides are straight lines, which are like the figure I draw for you - the two lines AB and AG and its arc is BDG. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|קו <big>משולש</big> אשר תושבתו עקמימות ב"ג וב' צלעיו הם קוים ישרים והם כצורה אשר אני מצייר לך ב' קוים א"ב וא"ג והתושבת קו עקום אשר עליו בד"ג{{#annotend:Eekc}} |
|- | |- | ||
| | | | ||
| − | : | + | :If you draw such a figure, as line BG, you have triangle ABG on it and arc BDG, and this is a segment of a circle. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואם אתה עושה כצורה הזאת קו כגון קו ב"ג יהיה לך משולש ישר אשר עליו אב"ג וצורת העקום אשר עליה בד"ג והיא שבר עגולה |
|- | |- | ||
| | | | ||
| − | :: | + | :[[File:Kelalim ba-Mispar 106.png|thumb|135px|left]] |
| − | | | + | |[[File:כללים במספר - קו.png|thumb|120px]] |
|- | |- | ||
| | | | ||
| − | :[ | + | :You find the area of each by itself, then sum the two areas and this is the area of the [sector]. |
| − | | | + | :<math>\scriptstyle{\color{OliveGreen}{\rm{Area_{triangle}+Area_{segment\ of\ circle}}}}</math> |
| + | |style="text-align:right;"|ואתה מרבע כל אחד מהם לבד ותאסוף שני המרובעים ויהיו תשבורת הצורה | ||
|- | |- | ||
| | | | ||
| − | + | ==== <span style=color:green>Rectangle</span> ==== | |
| − | + | ||
| − | + | | | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :{{#annot:rectangle|680|PLOt}}107) A rectangle whose parallel sides are identical i.e. both longitudinal lines are identical and both latitudinal lines are identical and all the angles are right angles. |
| − | + | :You want to know its area. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|קז <big>המרובע</big> ארוך שלא תהיינה צלעותיו שוות זו לזו אבל הנכוחים לבד הם שוות דהיינו שיהיו שני הארוכים שוים ושני הרחבים שוים וכל הזוויות הם נצבות<br> |
| − | + | ותרצה לידע תשברתו{{#annotend:PLOt}} | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :Multiply the side of the length by the side of the width and the result is its area. |
| − | + | :<math>\scriptstyle{\color{OliveGreen}{\rm{length_{rectangle}\times breadth_{rectangle}}}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|תרבה צלע האורך עם צלע הרוחב וכאשר יעלה כן יהיה {{#annot:term|816,1244|a5B3}}תשברתו{{#annotend:a5B3}} |
| − | |||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | *{{#annot:rectangle|680|OQC7}}Example: rectangle, its two long sides are 9 cubits and its two short sides are 5 cubits |
| + | |style="text-align:right;"|כגון מרובע ארוך ששתי צלעותיו האורך כל אחד מהם ט' אמות ושתי צלעות הרוחב כל אחד מהם ה' אמות כזה{{#annotend:OQC7}} | ||
|- | |- | ||
| | | | ||
| − | :[[File:Kelalim ba-Mispar | + | :[[File:Kelalim ba-Mispar 107.png|thumb|170px|left]] |
| − | |[[File:כללים במספר - | + | |[[File:כללים במספר - קז.png|thumb|200px]] |
|- | |- | ||
| | | | ||
| − | :the | + | :Multiply the length by the width and say: 5 by 9 is 45 and this is the measure of its area. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{5\sdot9=45}}</math> |
| + | |style="text-align:right;"|תרבה האורך עם הרוחב ואמור ה' ט' הם מ"ה וכן יהיה מניין תשברתו וכה"ל | ||
|- | |- | ||
| | | | ||
| − | + | ==== <span style=color:green>Rhombus</span> ==== | |
| − | + | ||
| − | + | | | |
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :{{#annot:rhombus|680|5zQq}}108) An equilateral quadrangle whose angles are not right angles is called a rhombus quadrangle. |
| − | |style="text-align:right;"| | + | :For example: rhombus, each of its sides is 10 cubits, its angles are not right angles, and its diagonals are not identical e.g.- its one diagonal is 12 cubits and the other diagonal is 16 cubits. |
| − | + | :You want to know its area. | |
| − | + | |style="text-align:right;"|קח <big>המרובע</big> שהוא שוה הצלעות ואינו נצב הזוויות והוא הנקרא ''{{#annot:term|1095,1526|6VRn}}מרובע המעויין{{#annotend:6VRn}}''<br> | |
| + | כגון מרובע שיש בכל צלעותיו י' אמות ואינו נצב הזוויות ואתה מוצא אלכסונותיו שאינן שוים כגון שיהיה האלכסון האחד י"ב אמות ואלכסון השני י"ו אמות<br> | ||
| + | ותרצה לידע תשברתו{{#annotend:5zQq}} | ||
|- | |- | ||
| | | | ||
| − | :: | + | :[[File:Kelalim ba-Mispar 108.png|thumb|90px|left]] |
| − | | | + | |[[File:כללים במספר - קח.png|thumb|90px]] |
| − | | | ||
| − | |||
|- | |- | ||
| − | |||
| | | | ||
| + | :Multiply one diagonal by half the other diagonal. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\rm{diagonal_1}\sdot\left(\frac{1}{2}\sdot \rm{diagonal_2}\right)}}</math> | ||
| + | |style="text-align:right;"|תמנה האלכסון האחד במחצית האלכסון השני | ||
|- | |- | ||
| | | | ||
| − | : | + | :Say: 16 times 6 is 96 and this is the measure of its area. |
| − | : | + | :<math>\scriptstyle{\color{blue}{16\sdot\left(\frac{1}{2}\sdot12\right)=16\sdot6=96}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואמור י"ו פעמי' ו' הם צ"ו וכן יהיה מנין תשברתו |
|- | |- | ||
| | | | ||
| − | : | + | :Or, say: 12 times 8 is 96 and this is the measure of its area. |
| − | | | + | :<math>\scriptstyle{\color{blue}{12\sdot\left(\frac{1}{2}\sdot16\right)=12\sdot8=96}}</math> |
| + | |style="text-align:right;"|או אמור י"ב פעמ' ח' צ"ו וכן יהיה מניין תשברתו וזו היא הצורה | ||
|- | |- | ||
| | | | ||
| − | + | ==== <span style=color:green>Equilateral Triangle</span> ==== | |
| − | + | ||
| − | |||
| | | | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| − | |||
| | | | ||
| + | :{{#annot:equilateral triangle|680|LpYW}}109) This shape is an equilateral triangle and you want to know its area | ||
| + | |style="text-align:right;"|קט ''{{#annot:term|595,1863|sp6o}}<big>משולש</big> שוה הצלעות{{#annotend:sp6o}}'' ותרצה לדעת תשברתו{{#annotend:LpYW}} | ||
|- | |- | ||
| | | | ||
| − | : | + | :[[File:Kelalim ba-Mispar 109.png|thumb|150px|left]] |
| − | | | + | |[[File:כללים במספר - קט.png|thumb|175px]] |
|- | |- | ||
| | | | ||
| − | : | + | :Know that its area is a third of the square of its side and a tenth of the square of the side. |
| − | + | :<math>\scriptstyle{\color{OliveGreen}{\left(\frac{1}{3}\sdot side^2\right)+\left(\frac{1}{10}\sdot side^2\right)}}</math> | |
| − | + | |style="text-align:right;"|דע כי תשברתו הוא שלישית מרובע צלעו ועשירית מרובע הצלע | |
| − | |||
| − | |||
| − | :<math>\scriptstyle | ||
| − | |style="text-align:right;"|הוא | ||
|- | |- | ||
| | | | ||
| − | + | *{{#annot:equilateral triangle|680|UGRq}}As the triangle, each of its sides is 15. | |
| − | + | |style="text-align:right;"|כגון משולש שכל אחד מצלעיו ט"ו{{#annotend:UGRq}} | |
| − | |style="text-align:right;"| | ||
| − | |||
|- | |- | ||
| − | |||
| | | | ||
| + | :Multiply one of its side and say: 15 times 15 is 225. | ||
| + | |style="text-align:right;"|תרבה אחת מצלעיו ואמור ט"ו פעמי' ט"ו הם רכ"ה | ||
|- | |- | ||
| | | | ||
| − | : | + | :Take a third of 225, which is 75; and a tenth of 225, which is 22 and a half; the total is 97 and a half and this is the area of the triangle. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|קח שלישית רכ"ה שהוא ע"ה ועשירית רכ"ה שהוא כ"ב וחצי ויהיה הכל צ"ז וחצי וכן יהיה תשבורת זה המשולש וכה"ל וזאת היא הצורה |
|- | |- | ||
| − | | | + | |colspan=2| |
| − | + | :<math>\scriptstyle{\color{blue}{\left(\frac{1}{3}\sdot15^2\right)+\left(\frac{1}{10}\sdot15^2\right)=\left(\frac{1}{3}\sdot225\right)+\left(\frac{1}{10}\sdot225\right)=75+\left(22+\frac{1}{2}\right)=97+\frac{1}{2}}}</math> | |
| − | |||
| − | | | ||
| − | |||
| − | |||
| − | :<math>\scriptstyle\ | ||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :If you want to know the area of the triangle in another way: |
| − | + | |style="text-align:right;"|ואם תרצה לדעת תשבורת המשולש בעניין אחר | |
| − | |style="text-align:right;"| | ||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| − | |||
| | | | ||
| + | :Multiply its height by half its base; or multiply its base by half the height. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\rm{height}\sdot\left(\frac{1}{2}\sdot\rm{side}\right)=\rm{side}\sdot\left(\frac{1}{2}\sdot\rm{height}\right)}}</math> | ||
| + | |style="text-align:right;"|תרבע העמוד ‫<ref>110r</ref>היוצא בו במחצית התושבת או תרבע התושבת במחצית העמוד | ||
|- | |- | ||
| | | | ||
| − | + | *{{#annot:equilateral triangle|680|m1Vo}}As the triangle, each of its sides is 15 and its height is 13. Like this: | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כגון משולש שכל אחד מצלעותיו ט"ו ועמודו י"ג כזה{{#annotend:m1Vo}} |
|- | |- | ||
| | | | ||
| − | : | + | :Multiply the height, which is 13, by half its base, which is 7 and a half. Say: 13 times 7 and a half is 97 and a half and this is the area of the triangle. |
| − | | | + | :<math>\scriptstyle{\color{blue}{13\sdot\left(\frac{1}{2}\sdot15\right)=13\sdot\left(7+\frac{1}{2}\right)=97+\frac{1}{2}}}</math> |
| + | |style="text-align:right;"|תרבה העמוד שהוא י"ג במחצית התושבת שהוא ז' וחצי ואמור י"ג פעמ' ז' וחצי הם צ"ז וחצי וכן יהיה תשבורת המשולש | ||
|- | |- | ||
| − | |||
| | | | ||
| + | :Or, multiply its base, which is 15, by half the height, which is 6 and a half. Say: 15 times 6 and a half is 97 and a half and this is its area. | ||
| + | :<math>\scriptstyle{\color{blue}{15\sdot\left(\frac{1}{2}\sdot13\right)=15\sdot\left(6+\frac{1}{2}\right)=97+\frac{1}{2}}}</math> | ||
| + | |style="text-align:right;"|או תרבע התושבת שהוא ט"ו במחצית העמוד שהוא ו' וחצי ואמור ט"ו פעמ' ו' וחצי צ"ז וחצי וכן יהיה תשברתו וכה"ל | ||
|- | |- | ||
| − | |||
| | | | ||
| − | + | ||
| + | === <span style=color:green>Equilateral Triangle</span> === | ||
| + | |||
| | | | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| + | !<span style=color:green>Figure Problem - height; Area - Equilateral Triangle</span> | ||
| | | | ||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :{{#annot:equilateral triangle|680,685|phM5}}110) If you want to know the area and the height of the triangle that is an equilateral triangle, do as follows: |
| − | + | :For example: triangle, each of its sides is 10. | |
| + | |style="text-align:right;"|קי <big>ואם</big> תרצה לדעת שבור העמוד מן המשולש שהוא שוה הצלעות עשה כענין זה<br> | ||
| + | כגון משולש שכל אחד מצלעותיו י' כזה{{#annotend:phM5}} | ||
|- | |- | ||
| | | | ||
| − | :: | + | :[[File:Kelalim ba-Mispar 110.png|thumb|150px|left]] |
| − | + | |[[File:כללים במספר - קי.png|thumb|170px]] | |
| − | |||
| − | | | ||
| − | | | ||
| − | |||
| − | | | ||
|- | |- | ||
| | | | ||
| − | : | + | :Multiply the side by itself. Say: 10 times 10 is 100. |
| − | : | + | |style="text-align:right;"|תרבה הצלע האחד בעצמו ואמור י' פעמ' י' הם ק‫' |
| − | |style="text-align:right;"| | ||
| − | |||
| − | |||
|- | |- | ||
| − | |||
| | | | ||
| + | :Multiply half its base, which is 5, by itself. Say: 5 times 5 is 25. | ||
| + | |style="text-align:right;"|ותרבה חצי התושבת שהוא ה' בעצמו ואמור ה' פעמ' ה' כ"ה | ||
|- | |- | ||
| | | | ||
| − | : | + | :Subtract it from 100; 75 remains. |
| − | + | |style="text-align:right;"|ותפחות אותם מק' ישארו ע"ה | |
| − | |style="text-align:right;"| | ||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :Extract the root of 75; it is approximately 8 and 2-thirds; and this is the size of the height. |
| − | | | + | |style="text-align:right;"|ותמצא הגדר של ע"ה והנו ח' וב' שלישי בקירוב והוא יהיה מספר העמוד |
|- | |- | ||
| − | | | + | |colspan=2| |
| − | + | :<math>\scriptstyle{\color{OliveGreen}{\sqrt{\rm{side}^2-\left(\frac{1}{2}\sdot\rm{side}\right)}}}{\color{blue}{=\sqrt{10^2-\left(\frac{1}{2}\sdot10\right)^2}=\sqrt{100-5^2}=\sqrt{100-25}=\sqrt{75}\approx8+\frac{2}{3}}}</math> | |
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :If you want to know the area of this triangle: |
| − | + | |style="text-align:right;"|ואם תרצה לדעת שברי זה המשולש | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |style="text-align:right;"| | ||
| − | |||
| − | ואם תרצה | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :Multiply the whole height by half its base, which is 5. |
| − | | | + | :<math>\scriptstyle{\color{OliveGreen}{\rm{height}\times\left(\frac{1}{2}\sdot\rm{side}\right)}}</math> |
| + | |style="text-align:right;"|תרבה כל העמוד עם חצי התושבת שהוא ה‫' | ||
|- | |- | ||
| | | | ||
| − | :: | + | :Or, multiply its whole base by half the height. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{OliveGreen}{\rm{side}\times\left(\frac{1}{2}\sdot\rm{height}\right)}}</math> |
| + | |style="text-align:right;"|או תרבה כל התושבת במחצית העמוד | ||
|- | |- | ||
| + | !<span style=color:green>Figure Problem - height; Area; Side - Equilateral Triangle</span> | ||
| | | | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| | | | ||
| + | :{{#annot:equilateral triangle|685|0JmD}}111) If you want to know the height of the triangle that is equilateral in another way. | ||
| + | |style="text-align:right;"|קיא <big>ואם</big> תרצה לדעת שיעור העמוד בעניין אחר מן המשולש שהוא שוה הצלעות{{#annotend:0JmD}} | ||
|- | |- | ||
| | | | ||
| − | :{{ | + | :Always subtract from the side of the equilateral triangle its 2 parts of 15 and this is the size of the height. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{OliveGreen}{\rm{side}-\left(\frac{2}{15}\sdot\rm{side}\right)}}</math> |
| − | והוא | + | |style="text-align:right;"|תפחות לעולם מן הצלע שלמשולש שוה הצלעות ב' חלקי' מט"ו והוא יהיה מספר העמוד |
|- | |- | ||
| | | | ||
| − | : | + | *{{#annot:equilateral triangle|680|JQy7}}Example: triangle, each of its sides is 15, you know that its height is 13, and you want to know its area. |
| − | + | |style="text-align:right;"|כגון משולש שכל אחד מצלעותיו [ט"ו]‫ | |
| − | + | הוי יודע כי עמודו י"ג<br> | |
| − | + | ואם תרצה לדעת תשברתו{{#annotend:JQy7}} | |
| − | |||
| − | |style="text-align:right;"|ואם תרצה לדעת | ||
|- | |- | ||
| | | | ||
| − | : | + | :Multiply 13, which is the height, by 7 and a half, which is half the base. |
| − | + | :<math>\scriptstyle{\color{blue}{13\sdot\left(\frac{1}{2}\sdot15\right)=13\sdot\left(7+\frac{1}{2}\right)}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|תרבה י"ג שהוא העמוד בז' וחצי שהוא חצי התושבת |
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | :: | + | :Or, the whole base, which is 15, by 6 and a half, which is half the size of the height. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{15\sdot\left(\frac{1}{2}\sdot13\right)=15\sdot\left(6+\frac{1}{2}\right)}}</math> |
| + | |style="text-align:right;"|או כל התושבת שהוא ט"ו בו' וחצי שהוא חצי השיעור מן העמוד | ||
|- | |- | ||
| | | | ||
| − | + | *{{#annot:equilateral triangle|679|KoRd}}If you know the height of the equilateral triangle and you want to know the side using the height. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואם תדע העמוד מן {{#annot:term|595,1863|Okmu}}המשולש השוה הצלעות{{#annotend:Okmu}} ותרצה לדעת הצלע מן העמוד ההוא{{#annotend:KoRd}} |
|- | |- | ||
| | | | ||
| − | : | + | :Multiply the height by itself. Add to this square its third, then find the root of the sum and this is the size of each side of the equilateral triangle. |
| − | + | :<math>\scriptstyle{\color{OliveGreen}{\sqrt{\rm{height}^2+\left[\frac{1}{3}\sdot\left(\rm{height}^2\right)\right]}}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|תרבע העמוד בעצמו והוסיף על זה המרובע שלישיתו ותידע כמה הוא גדר הכל והוא יהיה מדת כל צלע מצלעי המשולש שוה הצלעות |
|- | |- | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| | | | ||
| + | *{{#annot:equilateral triangle|679|DuKv}}Example: a triangle, whose height is 13, and you want to know its side. | ||
| + | |style="text-align:right;"|כגון משולש שהעמוד שלו הוא י"ג ותרצה לידע מידת הצלע{{#annotend:DuKv}} | ||
|- | |- | ||
| | | | ||
| − | : | + | :Multiply the height by itself and say: 13 times 13 is 169. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|תרבה העמוד בעצמו ואמור י"ג פעמ' י"ג קס"ט |
|- | |- | ||
| | | | ||
| − | + | :Add to it the third of 169, which is 56 and a third; it is 225 and a third. | |
| − | :the | + | |style="text-align:right;"|הוסף עליהם שלישית קס"ט שהם נ"ו ושליש יהיו רכ"ה ושליש |
| − | |style="text-align:right;"| | ||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Extract the root of 225 and a third, which is approximately 15 and this is the size of the side. |
| + | |style="text-align:right;"|תמצא הגדר של רכ"ה ושליש שהם ט"ו בקירוב והוא יהיה מספר הצלע | ||
|- | |- | ||
| − | | | + | |colspan=2| |
| − | :[ | + | :<math>\scriptstyle{\color{blue}{\sqrt{13^2+\left[\frac{1}{3}\sdot\left(13^2\right)\right]}=\sqrt{169+\left(\frac{1}{3}\sdot169\right)}=\sqrt{169+\left(56+\frac{1}{3}\right)}=\sqrt{225+\frac{1}{3}}\approx15}}</math> |
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | |
| − | + | === <span style=color:green>Scalene Triangle</span> === | |
| − | + | ||
| | | | ||
| − | |||
| − | |||
|- | |- | ||
| + | !<span style=color:green>Figure Problem - Side - Scalene Triangle</span> | ||
| | | | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | + | :{{#annot:scalene triangle|679|mpbY}}112) If a man tells you: a scalene triangle, its height is 12, one of its legs is 13 and the other is 15. | |
| − | : | + | :How much is the base? |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|קיב <big>אם</big> יאמר לך אדם ''{{#annot:triangle|596,1865|F605}}משולש מתחלף{{#annotend:F605}}'' עמודו י"ב והצלע האחר המקפת את ראשו י"ג והשנית ט"ו<br> |
| + | כמה תהיה התושבת{{#annotend:mpbY}} | ||
|- | |- | ||
| | | | ||
| − | :: | + | :The answer of this question: square one side, such as 13, whose square is 169. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והתשובה בשאלה הזאת תרבע הצלע האחת כגון י"ג שריבועה קס"ט |
|- | |- | ||
| | | | ||
| − | : | + | :Subtract from it the square of the height, which is 144; 25 remains, whose root is 5 and this is one segment of the base. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ותוציא ממנו מרובע העמוד והוא קמ"ד ישאר כ"ה שגדרו ה' והוא המעמד האחד |
|- | |- | ||
| | | | ||
| − | : | + | :Again, square the other side; its square is 225. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ושוב ורבע את הצלע השנית ומרובעה רכ"ה |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Subtract from it the square of the height; 81 remains, whose root is 9 and this is the second segment of the base. |
| + | |style="text-align:right;"|הוצא ממנה מרובע העמוד ישאר פ"א וגדרו ט' והוא המעמד השני | ||
|- | |- | ||
| − | |||
| | | | ||
| + | :The [sum of] these two segments is 14 and this is the length of the base. | ||
| + | |style="text-align:right;"|וב' המעמדי' האלה הם י"ד והוא אורך התושבת | ||
|- | |- | ||
| − | | | + | |colspan=2| |
| − | :{{ | + | :<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle{\color{OliveGreen}{\sqrt{\rm{side}_1^2-\rm{height}^2}+\sqrt{\rm{side}_2^2-\rm{height}^2}}}&\scriptstyle=\sqrt{13^2-12^2}+\sqrt{15^2-12^2}=\sqrt{169-144}+\sqrt{225-144}\\&\scriptstyle=\sqrt{25}+\sqrt{81}=5+9=14\\\end{align}}}</math> |
| − | |||
|- | |- | ||
| + | !<span style=color:green>Figure Problem - height - Scalene Triangle</span> | ||
| | | | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | :: | + | *{{#annot:scalene triangle|685|bJEk}}If you are told: a scalene triangle, the sum of its area with the height is 96, the base of the height is 14. |
| − | |style="text-align:right;"| | + | :How much is the height? |
| − | + | |style="text-align:right;"|ואם יאמר לך משולש מתחלף בתשברתו עם העמוד צ"ו ותושבת העמוד י"ד<br> | |
| + | כמה הוא העמוד{{#annotend:bJEk}} | ||
|- | |- | ||
| | | | ||
| − | :[ | + | :The answer: take half the base, always add one to it, then divide the number you have [= the sum of the area with the height] by it and you will find the height. |
| − | | | + | :<math>\scriptstyle{\color{OliveGreen}{\frac{\rm{Area+height}}{\left(\frac{1}{2}\sdot\rm{base}\right)+1}}}</math> |
| + | |style="text-align:right;"|תשובה קח מחצית התושבת והוסף אחד לעולם וחלק עליה את המספר אשר בידך ותמצא העמוד | ||
|- | |- | ||
| | | | ||
| − | ::<math>{\color{blue}{\ | + | :As when you divide the base of this question in two. Add one to its half; it is 8. Divide 96 by it; it is 12 and this is the length of the height. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{\frac{96}{\left(\frac{1}{2}\sdot14\right)+1}=\frac{96}{8}=12}}</math> |
| + | |style="text-align:right;"|כאילו היית מחלק בשאלה הזאת התושבת לשנים ומחציתה הוסף עליה אחד יהיו ח‫'<br> | ||
| + | חלק עליהם צ"ו יהיה י"ב והוא אורך העמוד | ||
|- | |- | ||
| | | | ||
| − | : | + | |
| − | | | + | === <span style=color:green>Isosceles Triangle</span> === |
| + | |||
| + | | | ||
|- | |- | ||
| − | + | !<span style=color:green>Figure Problem - Area; height; Side - Isosceles Triangle</span> | |
| | | | ||
|- | |- | ||
| | | | ||
| − | : | + | :{{#annot:isosceles triangle|680|9AmQ}}113) An isosceles triangle is a triangle whose legs are equal but the base is not equal to the legs. |
| − | |style="text-align:right;"| | + | :For example: this triangle, whose both legs are 15, and its base is 18. |
| + | :If we want to know its area. | ||
| + | |style="text-align:right;"|קיג ''{{#annot:triangle|597,2202|SINi}}<big>משולש</big> שוה השוקים{{#annotend:SINi}}'' והוא משולש ששוקיו שוים והתושבת אינו שוה עמהם<br> | ||
| + | ‫<ref>110v</ref>כגון זה המשולש שכל אחד משוקיו ט"ו והתושבת היא י"ח<br> | ||
| + | ואם נרצה לדעת שבריו{{#annotend:9AmQ}} | ||
|- | |- | ||
| | | | ||
| − | + | :[[File:Kelalim ba-Mispar 113.png|thumb|200px|left]] | |
| − | + | |[[File:כללים במספר - קיג.png|thumb|200px]] | |
| − | | | ||
| − | |||
| − | | | ||
| − | |||
| − | | | ||
|- | |- | ||
| | | | ||
| − | : | + | :First, we should find the size of its height and we do it this way: |
| − | + | |style="text-align:right;"|נדע מספר העמוד תחלה ונעשה בענין זה | |
| − | |style="text-align:right;"| | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | + | :We multiply one of the legs by itself. Say: 15 times 15 is 225. | |
| − | : | + | |style="text-align:right;"|נרבע אחד מן השוקים בעצמו ואמור ט"ו פעמי' ט"ו הם רכ"ה |
| − | |style="text-align:right;"| | ||
| − | |||
|- | |- | ||
| | | | ||
| − | :: | + | :We subtract the square of half the base from 225: half the base is 9; its square is 81; we subtract it from 225; 144 remains. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ונפחות מרכ"ה מרובע חצי התושבת וחצי התושבת הוא ט' ומרובעו פ"א ונפחות אותם מרכ"ה ישארו קמ"ד |
|- | |- | ||
| | | | ||
| − | + | :Extract the root of 144; it is 12. So, the size of this height is 12. | |
| − | + | |style="text-align:right;"|ותמצא השורש מקמ"ד והוא י"ב וכן יהיה מספר העמוד הזה י"ב | |
| − | |style="text-align:right;"| | ||
| − | |||
|- | |- | ||
| − | | | + | |colspan=2| |
| − | + | :<math>\scriptstyle{\color{OliveGreen}{\sqrt{\rm{side}^2-\left(\frac{1}{2}\sdot\rm{base}\right)^2}}}{\color{blue}{=\sqrt{15^2-\left(\frac{1}{2}\sdot18\right)^2}=\sqrt{225-9^2}=\sqrt{225-81}=\sqrt{144}=12}}</math> | |
| − | |||
|- | |- | ||
| | | | ||
| − | :: | + | :If you want to know the area of this triangle: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואם תרצה לדעת תשבורת המשולש הזה |
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{ | + | :Multiply the whole height, which is 12, by half the base, which is 9. Say: 9 times 12 is 108 and this is the area of this triangle. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{OliveGreen}{\rm{height}\times\left(\frac{1}{2}\sdot\rm{base}\right)}}{\color{blue}{=12\sdot\left(\frac{1}{2}\sdot18\right)=12\sdot9=108}}</math> |
| + | |style="text-align:right;"|תרבה כל העמוד שהוא י"ב על חצי התושבת שהוא ט' ואמור ט' פעמ' י"ב הוא ק"ח והוא תשבורת זה המשולש | ||
|- | |- | ||
| − | |||
| | | | ||
| − | + | :Or, multiply the whole base, which is 18, by half the height, which is 6. Say: 6 times 18 is 108 and this is its area. | |
| + | :<math>\scriptstyle{\color{OliveGreen}{\rm{base}\times\left(\frac{1}{2}\sdot\rm{height}\right)}}{\color{blue}{=18\sdot\left(\frac{1}{2}\sdot12\right)=18\sdot6=108}}</math> | ||
| + | |style="text-align:right;"|או תרבה כל התושבת שהוא י"ח על חצי העמוד שהוא ו' ואמ' ו' פעמ' י"ח הוא ק"ח והוא יהיה תשברתו וכה"ל | ||
|- | |- | ||
| | | | ||
| − | + | *{{#annot:isosceles triangle|679|RGa1}}If you know the size of the height and the base of an isosceles triangle and you want to know the size of both legs. | |
| − | :You want to | + | :For example: this triangle, whose height is 12, and its base is 18. |
| − | |style="text-align:right;"| | + | :You want to know how much are the legs. |
| − | + | |style="text-align:right;"|ואם תדע מדת העמוד ומדת התושבת ממשולש שוה השוקים ותרצה לדעת מדת כל אחד מהשוקים<br> | |
| + | כגון זה המשולש שעמודו י"ב ותושבתו י"ח<br> | ||
| + | ותרצה לדעת מדת השוקים{{#annotend:RGa1}} | ||
|- | |- | ||
| | | | ||
| − | : | + | :Multiply the height, which is 12, by itself. Say: 12 times 12 is 144. |
| − | | | + | |style="text-align:right;"|תרבע העמוד בעצמו שהוא י"ב ואמור י"ב פעמ' י"ב הם קמ"ד |
|- | |- | ||
| | | | ||
| − | : | + | :Add to it the square of half the base, which is 9 and its square is 81; their sum is 225. |
| − | + | |style="text-align:right;"|הוסף עליהם מרובע חצי התושבת שהוא ט' שיבא פ"א וקבצם יהיו רכ"ה | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | : | + | :Extract the root of 225; it is 15 and this is the size of each of the legs. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|תמצא השורש מרכ"ה והוא ט"ו וכן יהיה מדת כל אחד מהשוקיים וכה"ל |
|- | |- | ||
| − | | | + | |colspan=2| |
| − | : | + | :<math>\scriptstyle{\color{OliveGreen}{\sqrt{\rm{height}^2+\left(\frac{1}{2}\sdot\rm{base}\right)^2}}}{\color{blue}{=\sqrt{12^2+\left(\frac{1}{2}\sdot18\right)^2}=\sqrt{144+9^2}=\sqrt{144+81}=\sqrt{225}=15}}</math> |
| − | |||
|- | |- | ||
| | | | ||
| − | + | ||
| − | : | + | === <span style=color:green>Area</span> === |
| − | | | + | |
| − | + | | | |
|- | |- | ||
| | | | ||
| − | : | + | ==== <span style=color:green>Scalene Triangle</span> ==== |
| − | + | ||
| − | + | | | |
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :{{#annot:scalene triangle|680|dbrx}}114) A scalene triangle such as triangle ABG: AB is 13, BG is 14, and AG is 15 |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|קיד ''{{#annot:triangle|596,1865|Hcvc}}<big>משולש</big> מתחלף הצלעות{{#annotend:Hcvc}}'' כגון משולש אב"ג שצלע א"ב י"ג וצלע ב"ג י"ד וצלע א"ג ט"ו{{#annotend:dbrx}} |
|- | |- | ||
| | | | ||
| − | : | + | :[[File:Kelalim ba-Mispar 114.png|thumb|200px|left]] |
| − | | | + | |[[File:כללים במספר - קיד.png|thumb|200px]] |
|- | |- | ||
| | | | ||
| − | : | + | :We can find the area of this triangle only by using the height. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וזה המשולש לא נוכל לדעת תשברתו אלא מן העמוד |
| − | |||
|- | |- | ||
| + | |<span style=color:red>[Although later on (problem 116) a general formula is given for finding the area of any triangle according to its three sides without needing to know the height]</span> | ||
| | | | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|כי | + | :Because, for every triangle, whether equilateral, or scalene, its area is the product of its height by half the base, or the product of the whole base by half the height. |
| − | + | |style="text-align:right;"|כי כל משולש בין שוה הצלעות בין מתחלף הצלעות תשברתו הוא ברבוע העמוד על חצי התושבת או בריבוע כל התושבת בחצי העמוד | |
| − | + | |- | |
| − | + | |colspan=2| | |
| + | :<math>\scriptstyle{\color{OliveGreen}{\rm{height}\times\left(\frac{1}{2}\sdot\rm{base}\right)=\rm{base}\times\left(\frac{1}{2}\sdot\rm{height}\right)}}</math> | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | :Therefore, we have to find the height and the segments it defines on the base. |
| − | + | |style="text-align:right;"|ולכן נצטרך להוציא העמוד ולהגביל מעמדו בתושבת | |
|- | |- | ||
| − | |||
| | | | ||
| + | :Because, the height of this triangle does not stand on half its base, but is closer to one side [than the other]. | ||
| + | |style="text-align:right;"|כי העמוד במשולש הזה אינו עומד במחצית התושבת אבל נוטה ממחצית התושבת אל צד אחד | ||
|- | |- | ||
| | | | ||
| − | : | + | :We call the long segment of the base from its meeting point with the height "the long segment" and the other "the short segment". |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והצד הארוך מגבול מעמדו אנו קוראים לו ''מעמד ארוך'' והצד השני ''מעמד קצר'' |
| − | |||
| − | |||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | :If we want to find the long segment: |
| + | |style="text-align:right;"|ואם נרצה להוציא המעמד הארוך | ||
|- | |- | ||
| − | |the | + | | |
| − | |style="text-align:right;"| | + | :We take the square of one of the two sides, between which the height is drawn; this is side AG, which is 15; its square is 225. |
| + | |style="text-align:right;"|נקח מרובע הצלע משתי הצלעות אשר העמוד יוצא מבניהם והוא צלע א"ג שהוא ט"ו ומרבעו רכ"ה | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | :We add this square to the square of the base, which is 14 and its square is 196; the [sum of the] two squares is 421. |
| + | |style="text-align:right;"|ונחבר המרובע הזה אל מרובע התושבת שהוא י"ד ומרובעו קצ"ו<br> | ||
| + | ויהיו שני המרובעים תכ"א | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | :We subtract from it the square of the shorter side, which is side AB that is 13 and its square is 169; 252 remains. |
| + | |style="text-align:right;"|נוציא מהם מרובע צלע הקצר שהוא צלע א"ב שהוא י"ג ומרובעו קס"ט וישארו רנ"ב | ||
|- | |- | ||
| − | |the | + | | |
| − | |style="text-align:right;"| | + | :We divide 252 in two; the half is 126. |
| − | + | |style="text-align:right;"|נחלק רנ"ב לשנים ויהיה המחצית קכ"ו | |
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | :We divide 126 by the base, which is 14; it is 9 and this is [the long segment of the base], for line GD is 9. |
| + | |style="text-align:right;"|נחלק קכ"ו על התושבת שהוא י"ד יהיו ט' והוא מרחק גבול מעמד העמוד מן הצלע הארוך הרי שקו ג"ד הוא ט‫' | ||
|- | |- | ||
| − | | | + | |colspan=2| |
| − | + | :<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle\frac{\frac{1}{2}\sdot\left[\left(AG^2+BG^2\right)-AB^2\right]}{BG}&\scriptstyle=\frac{\frac{1}{2}\sdot\left[\left(15^2+14^2\right)-13^2\right]}{14}=\frac{\frac{1}{2}\sdot\left[\left(225+196\right)-169\right]}{14}=\frac{\frac{1}{2}\sdot\left(421-169\right)}{14}\\&\scriptstyle=\frac{\frac{1}{2}\sdot252}{14}=\frac{126}{14}=9\\\end{align}}}</math> | |
| − | |||
|- | |- | ||
| | | | ||
| − | :: | + | :If we want to find the short segment: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואם נרצה לדעת המעמד הקצר |
|- | |- | ||
| | | | ||
| − | : | + | :We take the square of short side, which is 13; its square is 169. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|נקח מרובע הצלע הקצר שהוא י"ג ומרובעו קס"ט |
|- | |- | ||
| | | | ||
| − | :[ | + | :With the square of the base, which is 196; the [sum of the] two squares is 365. |
| − | | | + | |style="text-align:right;"|עם מרובע התושבת שהוא קצ"ו ויהיו שני המרובעי' שס"ה |
|- | |- | ||
| | | | ||
| − | : | + | :We subtract 225 from it, which is the square of line AG that is the long side and it is 15; 140 remains. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|נוציא מהם רכ"ה שהוא מרובע קו א"ג שהוא הצלע הארוך שהוא ט"ו ישארו ק"מ |
|- | |- | ||
| | | | ||
| − | : | + | :Divide it in two; the half is 70. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|חלקם לשנים ויהיה המחצית ע‫' |
|- | |- | ||
| | | | ||
| − | : | + | :We divide it by the base, which is 14; it is 5 and this is [the short segment of the base]. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|נחלקם על התושבת שהוא י"ד יהיו ה' וכן מרחק מעמד העמוד מן הצלע הקצר |
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle\frac{\frac{1}{2}\sdot\left[\left(AB^2+BG^2\right)-AG^2\right]}{BG}&\scriptstyle=\frac{\frac{1}{2}\sdot\left[\left(13^2+14^2\right)-15^2\right]}{14}=\frac{\frac{1}{2}\sdot\left[\left(169+196\right)-225\right]}{14}=\frac{\frac{1}{2}\sdot\left(365-225\right)}{14}\\&\scriptstyle=\frac{\frac{1}{2}\sdot140}{14}=\frac{70}{14}=5\\\end{align}}}</math> | ||
|- | |- | ||
| | | | ||
| − | : | + | :If you want to know the length of the height: |
| − | : | + | |style="text-align:right;"|ואם תרצה לדעת אורך זה העמוד |
| − | |style="text-align:right;"| | ||
| − | ואם | ||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :We square the side and subtract from its square the square of the segment that is closer to it. Then, we extract the root of the remainder and this is the length of the height. |
| − | :: | + | |style="text-align:right;"|נרבע הצלע ונוציא ממרובעו מרובע המעמד הדבק בו ונקח גדר הנשאר והוא יהיה אורך העמוד |
| − | + | |- | |
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\sqrt{side_1^2-\left(\rm{long\ segment}\right)^2}=\sqrt{side_2^2-\left(\rm{short\ segment}\right)^2}}}</math> | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Example: we square the short side, which is 13 in this triangle; it is 169. |
| + | |style="text-align:right;"|המשל בזה נרבע הצלע הקצר שהוא י"ג בזה המשולש יהיו קס"ט | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | :We subtract from 169 the square of the short segment attached to it, i.e. line DB, which is 5 and its square is 25; 144 remains. |
| + | |style="text-align:right;"|נפחות מקס"ט מרובע המעמד הקצר הדבק בו דהיינו קו ד"ב שהוא ה' ומרובעו כ"ה ישארו קמ"ד | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | :The root of 144 is 12, so the size of the height is 12. |
| + | |style="text-align:right;"|וגדר קמ"ד הוא י"ב ‫<ref>111r</ref>הרי שמספר העמוד הוא י"ב | ||
|- | |- | ||
| − | | | + | |colspan=2| |
| − | + | :<math>\scriptstyle{\color{blue}{\sqrt{AB^2-DB^2}=\sqrt{13^2-5^2}=\sqrt{169-25}=\sqrt{144}=12}}</math> | |
|- | |- | ||
| | | | ||
| − | : | + | :Likewise, if we square the long side, which is 15 and its square is 255. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכן אם נרבע הצלע הארוך שהוא ט"ו ומרובעו רכ"ה |
|- | |- | ||
| | | | ||
| − | : | + | :We subtract from it the square of the long segment, i.e. line GD, which is 9 and its square is 81; 144 remains, as remained from the short side. |
| − | + | |style="text-align:right;"|ונפחות ממנו מרובע המעמד הארוך דהיינו קו ג"ד שהוא ט' ומרובעו פ"א ישארו קמ"ד כמו שנשאר מן הצלע הקצר | |
| − | |||
| − | |||
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | :The root of 144 is 12, and this is the length of the height. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וגדר קמ"ד הוא י"ב והוא אורך העמוד |
|- | |- | ||
| − | | | + | |colspan=2| |
| − | + | :<math>\scriptstyle{\color{blue}{\sqrt{AG^2-GD^2}=\sqrt{15^2-9^2}=\sqrt{225-81}=\sqrt{144}=12}}</math> | |
|- | |- | ||
| − | |||
| | | | ||
| + | :If you want to know the area of this triangle: | ||
| + | |style="text-align:right;"|ואם תרצה לדעת תשבורת זה המשולש | ||
|- | |- | ||
| | | | ||
| − | :{{ | + | :Multiply the whole height, which is 12, by half the base, which is 7; the result is 84 and this is its area. |
| − | + | :<math>\scriptstyle{\color{OliveGreen}{\rm{height}\times\left(\frac{1}{2}\sdot\rm{base}\right)}}{\color{blue}{=12\sdot\left(\frac{1}{2}\sdot14\right)=12\sdot7=84}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|תרבה כל העמוד שהוא י"ב בחצי התושבת שהוא ז' שיבואו פ"ד והוא תשברתו |
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :Or, multiply the whole base, which is 14, by half the height, which is 6; it is 84 and this is its area. |
| − | | | + | :<math>\scriptstyle{\color{OliveGreen}{\rm{base}\times\left(\frac{1}{2}\sdot\rm{height}\right)}}{\color{blue}{=14\sdot\left(\frac{1}{2}\sdot12\right)=14\sdot6=84}}</math> |
| + | |style="text-align:right;"|או תרבה כל התושבת שהוא י"ד בחצי העמוד שהוא ו' ויהיו פ"ד והוא תשברתו וכה"ל וזאת היא צורתה | ||
|- | |- | ||
| | | | ||
| − | + | ||
| − | + | ==== <span style=color:green>Right Triangle</span> ==== | |
| − | + | ||
| − | + | | | |
| − | |||
| − | |||
|- | |- | ||
| − | | | + | | |
| + | :{{#annot:right triangle|680|tu7r}}115) This is the shape of the triangle that is called a right-angled triangle. | ||
| + | :If you want to know its area: | ||
| + | |style="text-align:right;"|קטו <big>המשולש</big> הזה נקרא ''{{#annot:triangle|1104,1341|c8w5}}משולש נצב הזויות{{#annotend:c8w5}}''<br> | ||
| + | ואם תרצה לדעת תשברתו{{#annotend:tu7r}} | ||
|- | |- | ||
| | | | ||
| − | :{{ | + | :Multiply one of the perpendicular sides by half the other side. |
| − | + | :<math>\scriptstyle{\color{OliveGreen}{base_1\times\left(\frac{1}{2}\sdot base_2\right)}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|תרבה הצלע האחד מן הצלעות המקיפות בזוית הנצבה בחצי הצלע האחרת |
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | *{{#annot:right triangle|680|JaBk}}Example: if line BA is 10 and line BG is also 10. |
| − | | | + | |style="text-align:right;"|כגון אם היה קו ב"א י' וקו ב"ג ג"כ י‫'{{#annotend:JaBk}} |
|- | |- | ||
| − | | | + | | |
| − | | | + | :[[File:Kelalim ba-Mispar 115.png|thumb|200px|left]] |
| + | |[[File:כללים במספר - קטו.png|thumb|200px]] | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | :Say: 10 times 5 is 50 and this is its area. |
| + | :<math>\scriptstyle{\color{blue}{10\times\left(\frac{1}{2}\sdot10\right)=10\sdot5=50}}</math> | ||
| + | |style="text-align:right;"|אמור י' פעמ' ה' הם נ' והוא תשברתו | ||
|- | |- | ||
| − | | | + | | |
| − | + | :Because you see that it is half a square. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כי אתה רואה שהוא חצי מרובע שוה הצלעות נצב הזוויות |
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :If it were a whole square, we would have say 10 times 10 is 100. |
| − | |style="text-align:right;"|ואם | + | |style="text-align:right;"|ואם היה מרובע שלם היינו אומרי' י' פעמ' י' הם ק‫' |
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | :: | + | :Now that it is half a square, we say: 10 times 5 is 50. The same for any similar calculation. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ועכשיו שהוא חצי מרובע נאמר י' פעמ' ה' הם נ' וכן לכל חשבון כזה |
|- | |- | ||
| − | | | + | |colspan=2| |
| − | + | :<math>\scriptstyle{\color{blue}{10\times\left(\frac{1}{2}\sdot10\right)=10\sdot5=50=\frac{1}{2}\sdot100=\frac{1}{2}\sdot10^2}}</math> | |
|- | |- | ||
| | | | ||
| − | : | + | ==== <span style=color:green>Heron's Theorem</span> ==== |
| + | |||
| | | | ||
|- | |- | ||
| − | | | + | | |
| − | + | :116) General Rule: to know the area of a triangle without considering the height. This calculation is called "the calculation of differences" <span style=color:green>[= '''Heron's Theorem''']</span> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|קיו <big>כלל</big> גדול לדעת תשבורת המשולש שאינך צריך בו אל הוצאת העמוד והחשבון הזה נקרא ''חשבון המותרות'' |
| − | |||
| − | |||
|- | |- | ||
| − | | | + | | |
| − | + | :Know the half of each of the triangle's sides and sum all these halves. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והוא שתדע מחצית כל אחד מצלעי המשולש ותקבץ כל המחציות האלה |
| − | |||
| − | והוא | ||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | | |
| − | + | :Know the excess of their sum over each of the sides and keep these excesses. | |
| − | + | |style="text-align:right;"|ותדע מותר כללם על כל צלע וצלע ותשמור המותרות האלה | |
| − | |style="text-align:right;"| | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Multiply one by the other, then multiply the resulting product by the third number. |
| − | + | |style="text-align:right;"|ותמנה אחד מהם בשיני והמספר הנקבץ מנה אותו בשלישי במספר | |
| − | |||
|- | |- | ||
| − | |||
| | | | ||
| + | :Multiply what you receive from this calculation by the total sum of the halves. | ||
| + | |style="text-align:right;"|ואשר יכנס בידך מהחשבון הזה מנה אותו בכל כלל המחציות אשר קבצת | ||
|- | |- | ||
| | | | ||
| − | : | + | :The resulting number is the square of the area of the triangle. |
| − | + | |style="text-align:right;"|ויהיה המספר הזה מרובע תשבורת המשולש | |
| − | |style="text-align:right;"| | ||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :If you will extract the root of this number you will find the area, |
| − | | | + | |style="text-align:right;"|ואם תוציא גדר המספר הזה תמצא התשבורת |
|- | |- | ||
| − | | | + | |colspan=2| |
| − | <math>\scriptstyle \ | + | :<math>\scriptstyle{\color{OliveGreen}{\begin{cases}\scriptstyle side_1=a\\\scriptstyle side_2=b\\\scriptstyle side_3=c\\\scriptstyle\frac{1}{2}a+\frac{1}{2}b+\frac{1}{2}c=s\end{cases}\scriptstyle\longrightarrow Area_{\triangle}=\sqrt{\left(s-a\right)\times\left(s-b\right)\times\left(s-c\right)\times s}}}</math> |
| − | |||
|- | |- | ||
| | | | ||
| − | + | *{{#annot:triangle|680|vlEX}}Example: a triangle whose one side is 10, the second is 8, and the third is 6 | |
| − | + | |style="text-align:right;"|המשל בזה משולש שצלעו האחד י' והשני ח' והשלישי ו‫'{{#annotend:vlEX}} | |
| − | |style="text-align:right;"| | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | :: | + | :[[File:Kelalim ba-Mispar 116.png|thumb|200px|left]] |
| − | : | + | |[[File:כללים במספר - קטז.png|thumb|200px]] |
| − | | | ||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :If you sum half these three sides, the sum is 12. |
| − | + | :<math>\scriptstyle{\color{blue}{\frac{1}{2}\sdot\left(10+8+6\right)=12}}</math> | |
| − | |style="text-align:right;"|ואם | + | |style="text-align:right;"|ואם אתה מקבץ מחצית ג' הצלעות האלה יהיה המספר י"ב |
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :The excess of 12 over one side is 2; over the second is 4; and over the third is 6. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ומותר י"ב על הצלע האחד ב' ועל השני ד' ועל השלישי ו‫' |
|- | |- | ||
| | | | ||
| − | : | + | :Multiply 2, which is the excess over one side, by 4, which is the excess over the second side; it is 8. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואתה בוא ומנה ב' שהוא מותר הצלע האחד בד' שהוא מותר הצלע השני יהיו ח‫' |
|- | |- | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| | | | ||
| + | :Multiply this 8 by 6, which is the excess over the third side; it is 48. | ||
| + | |style="text-align:right;"|בוא ומנה אלו הח' בו' שהוא מותר הצלע השלישי ויהיו מ"ח | ||
|- | |- | ||
| | | | ||
| − | : | + | :Multiply also 48 by 12, which is the total sum of the halves; it is 576; and 576 is the square of the area. |
| − | + | |style="text-align:right;"|שוב ומנה מ"ח בי"ב שהוא כלל המחציות ויהיו תקע"ו ותקע"ו הוא מרובע התשבורת | |
| − | |||
| − | |||
| − | |style="text-align:right;"| | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :When you extract the root of 576, it is 24 and it is the area of this triangle. |
| − | | | + | |style="text-align:right;"|ואם תמצא הגדר של תקע"ו יהיו כ"ד והוא יהיה תשבורת זה המשולש וכה"ל |
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |colspan=2| |
| − | + | :<math>\scriptstyle{\color{blue}{\sqrt{\left(12-10\right)\times\left(12-8\right)\times\left(12-6\right)\times12}=\sqrt{2\sdot4\sdot6\sdot12}=\sqrt{8\sdot6\sdot12}=\sqrt{48\sdot12}=\sqrt{576}=24}}</math> | |
|- | |- | ||
| − | | | + | | |
| − | + | ||
| − | + | ==== <span style=color:green>Parallelogram</span> ==== | |
| − | + | ||
| − | + | | | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | | | ||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :{{#annot:parallelogram|680|kPxn}}117) Quadrangle whose two long sides are equal, both are 25, its two short sides are equal, both are 15, and its angles are not right angles. |
| − | + | |style="text-align:right;"|קיז <big>מרובע</big> ששני אורכיו שוים וכל אחד מהם כ"ה ושני רחביו שוים וכל אחד מהם ט"ו ואין זוויותיו נצבות כגון זה{{#annotend:kPxn}} | |
| − | : | ||
| − | |style="text-align:right;"| | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | :: | + | :[[File:Kelalim ba-Mispar 117.png|thumb|200px|left]] |
| − | | | + | |[[File:כללים במספר - קיז.png|thumb|200px]] |
| − | | | ||
| − | | | ||
| − | | | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | :[ | + | :When we come to measure its area, we measure its diagonal AG; it is 20 and it cuts the quadrangle into these two [equal] triangles. We can find the area of this quadrangle only by finding the area of these two triangles. |
| − | | | + | |style="text-align:right;"|כשנבוא לרבעו נמדוד בו אלכסון א"ג והיה כ' אמה והוא החולק הזה המרובע לב' משולשים שוים ואין אנו יכולין לדעת שברי זה המרובע אלא מתוך ריבוע ב' המשולשים האלה |
|- | |- | ||
| | | | ||
| − | : | + | :Therefore, if we find the height of one of them, we know the area of that triangle; we double it, and by that we find the area of the two triangles, i.e. the area of the whole quadrangle. |
| − | + | :<math>\scriptstyle{\color{OliveGreen}{\rm{side}\times\rm{height}}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ומפני זה אם אנו מוציאים את העמוד באחד מהם נדע תשבורת המשולש ההוא ונכפול אותו ונמצא תשבורת שני המשולשים דהיינו תשבורת כל זה המרובע |
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :If you draw the height on side AB of triangle ABG, the height is 12 and the area of the triangle is 150. Double 150 is 300 and it is the area of the quadrangle. |
| − | + | :<math>\scriptstyle{\color{blue}{2\sdot\rm{Area_{\triangle_{ABG}}}=2\sdot150=300}}</math> | |
| − | |style="text-align:right;"|ואם | + | |style="text-align:right;"|ואם תוציא עמוד ‫<ref>111v</ref>במשולש אב"ג על צלע א"ב יהיה העמוד י"ב ותשבורת המשולש ק"נ וכפל ק"נ הוא ש' והוא תשבורת המרובע הזה |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :Anyone who does not understand this way, and multiply the length by the width, as done with the other quadrangles that have right angles, would say 25 times 15; the result is 375, then would see how much is the error between the two calculations, for the square exceeds. |
| − | | | + | :<math>\scriptstyle{\color{blue}{300\neq375=25\sdot15=side_1\times side_2}}</math> |
| + | |style="text-align:right;"|ומי שלא היה מבין זה הדרך והיה מרבע האורך עם הרוחב כשאר המרובעים שהם על זוויות נצבות והיה אומ' כ"ה פעמ' ט"ו היו עולים שע"ה ראה כמה טעות בין שני החשבונות המרובע עודף | ||
|- | |- | ||
| + | !<span style=color:green>Additional segment: the differences between the square and its inner circle and the inner square of the inner circle</span> | ||
| | | | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :The square exceeds its inner circle; the inner circle exceeds its inner square. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|המרובע עודף על העגול שבתוכו<br> |
| + | העגול עודף על המרובע שבתוכו | ||
|- | |- | ||
| | | | ||
| − | : | + | :<span style=color:blue>The external square exceeds its inner circle by <math>\scriptstyle{\color{blue}{\frac{3}{14}}}</math>; the inner circle exceeds its inner square by <math>\scriptstyle{\color{blue}{\frac{4}{14}}}</math>.</span> |
| − | + | |[[File:ריבוע בתוך עיגול בתוך ריבוע.png|thumb|200px]] | |
| − | | | ||
|- | |- | ||
| | | | ||
| − | + | ||
| + | ==== <span style=color:green>Trapezoid</span> ==== | ||
| + | |||
| + | | | ||
|- | |- | ||
| − | | | + | | |
| + | ===== <span style=color:green>Isosceles Trapezoid</span> ===== | ||
| + | |||
| | | | ||
|- | |- | ||
| | | | ||
| − | :{{#annot: | + | :{{#annot:isosceles trapezoid|680|jnJX}}118) A trapezoid such as this shape ABGD. |
| − | |style="text-align:right;"| | + | :AB and GD are equal, both 13. |
| + | :AG and BD are parallel and not equal to each other. | ||
| + | :AG is 8 cubits and BD is 18 cubits. | ||
| + | |style="text-align:right;"|קיח ''{{#annot:term|1094,2204|4hrz}}<big>קטומת</big> הראש{{#annotend:4hrz}}'' כגון זאת הצורה שיש לה ד' צלעות ועליהם אבג"ד<br> | ||
| + | וצלע א"ב וג"ד הם שוות וכל אחד מהם י"ג<br> | ||
| + | וצלעי א"ג וב"ד הם נכוחיים ואינם שוים זה לזה<br> | ||
| + | וצלע א"ג ח' אמות וצלע ב"ד י"ח אמות{{#annotend:jnJX}} | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | :This shape is called isosceles trapezoid. |
| + | |style="text-align:right;"|והצורה הזאת נקראת {{#annot:trapezoid|597,1247|s0uE}}קטומת הראש שוה{{#annotend:s0uE}} | ||
|- | |- | ||
| | | | ||
| − | : | + | :Side AG, which is the shorter of the two parallel sides is called "the upper base". |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וצלע א"ג שהיא הקצרה משתי צלעות הנכוחייות נקראת ''ראש הקטומה'' |
|- | |- | ||
| | | | ||
| − | : | + | :Side BD, which is the longer, is called the "lower base". |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וצלע ב"ד הארוכה נקראת ''תושבת הקטומה'' |
|- | |- | ||
| − | | | + | | |
| − | + | :[[File:Kelalim ba-Mispar 118-1.png|thumb|200px|left]] | |
| − | | | + | |[[File:כללים במספר - קיח -1.png|thumb|200px]] |
| − | | | ||
| − | | | ||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | + | :Whoever wants to measure this shape must first extract its height. | |
| − | + | |style="text-align:right;"|והרוצה למדוד הצורה הזאת יוציא {{#annot:term|1111,2206|N2oI}}עמודה{{#annotend:N2oI}} ראשונה | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | :The | + | :The way to extract it is to subtract the upper base of the trapezoid from its lower base. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ודרך הוצאתו הוא שיפחות ראש הקטומה משרשה מתושבתה |
|- | |- | ||
| | | | ||
| − | : | + | :To divide the difference between them by two. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והעודף ביניהם יחלק אותו לשנים |
|- | |- | ||
| | | | ||
| − | : | + | :To know the square of the half. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וידע מרובע המחצית |
|- | |- | ||
| | | | ||
| − | : | + | :To subtract from this square the square of one of the two equal sides. |
| − | + | |style="text-align:right;"|ויפחות מהמרובע הזה מרובע אחת מן הצלעות השוות | |
| − | |style="text-align:right;"| | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | :[[ | + | :Then, to know the root of the remainder and it is the size of the height. |
| − | | | + | :<math>\scriptstyle{\color{OliveGreen}{\sqrt{\rm{side}^2-\left[\frac{1}{2}\sdot\left[\left(\rm{bottom\ base}\right)-\left(\rm{upper\ base}\right)\right]\right]^2}}}</math> |
| + | |style="text-align:right;"|והנשאר ממרובע הצלע ידע שרשו והוא יהיה מספר העמוד | ||
|- | |- | ||
| | | | ||
| − | : | + | :In this shape, we subtract 8, which is the upper base of the trapezoid, from 18, which is its lower base; 10 remains. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ובצורה הזאת נפחות מנין ח' שהוא ראש הקטומה מן י"ח שהוא התושבת ישאר י‫' |
|- | |- | ||
| | | | ||
| − | : | + | :The half of 10 is 5 and this is [the length of] the excess segments: line BH from point H, and line DZ from point Z. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והמחצית מי' הוא ה' והוא יהיה ודאי חלק העודף האחד מנקודת ה' קו ב"ה ומנקודת ז' קו ד"ז |
|- | |- | ||
| | | | ||
| − | : | + | :We draw lines from A to H and from G to Z. It is known that each of these lines is perpendicular to base BD. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ונוציא קו מא' אל ה' ומן ג' אל ז' ובידוע שכל קו מהם הוא עמוד על תושבת ב"ד |
|- | |- | ||
| | | | ||
| − | : | + | :Triangle AHB is right angled and its hypotenuse is line AB. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ויהיה משולש אה"ב נצב הזוית ומיתר הזוית הזאת הוא קו א"ב |
|- | |- | ||
| | | | ||
| − | : | + | :It is known that the square of this hypotenuse, which is 13, is equal to [the sum of] the square of BH, which we know is 5, and the square of AH, which we want to know. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ובידוע כי מרובע המיתר הזה שהוא י"ג שוה למרובע ב"ה שהוא אשר ידענו שהוא ה' ולמרובע א"ה שאנו רוצים לדעתו |
|- | |- | ||
| | | | ||
| − | : | + | :If we subtract the square of 5, which is 25, from the square of 13, which is 169, 144 remains. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואם נפחות מרובע ה' שהוא כ"ה ממרובע י"ג שהוא קס"ט ישאר קמ"ד |
|- | |- | ||
| | | | ||
| − | : | + | :It is known that the root of 144, which is 12, is the size of the height. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ובידוע שגדר קמ"ד והוא י"ב יהיה מדת העמוד |
|- | |- | ||
| − | | | + | |colspan=2| |
| − | + | :<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle AH&\scriptstyle=\sqrt{AB^2-\left[\frac{1}{2}\sdot\left(BD-AG\right)\right]^2}=\sqrt{13^2-\left[\frac{1}{2}\sdot\left(18-8\right)\right]^2}=\sqrt{169-\left(\frac{1}{2}\sdot10\right)^2}\\&\scriptstyle=\sqrt{169-5^2}=\sqrt{169-25}=\sqrt{144}=12\\\end{align}}}</math> | |
| − | |||
| − | |||
|- | |- | ||
| − | | | + | | |
| − | + | :If you want to know the area of this shape: | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואם תרצה לדעת תשבורת זאת הצורה |
|- | |- | ||
| − | | | + | | |
| − | + | :Sum the upper base with the lower base; it is 26. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|תכניס הראש עם התושבת ויהיו כ"ו |
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | :Take its half; it is 13. |
| + | |style="text-align:right;"|קח מחציתם והם י"ג | ||
|- | |- | ||
| | | | ||
| − | + | :Multiply it by 12, which is the size of the height; it is 156 and this is its area. | |
| − | == | + | |style="text-align:right;"|ומנה אותם בי"ב שהוא מדת העמוד יהיו קנ"ו והוא תשברתו |
| − | + | |- | |
| − | + | |colspan=2| | |
| + | :<math>\scriptstyle{\color{OliveGreen}{\left[\frac{1}{2}\sdot\left[\left(\rm{top\ base}\right)+\left(\rm{bottom\ base}\right)\right]\right]\sdot\rm{height}}}{\color{blue}{=\left[\frac{1}{2}\sdot\left(8+18\right)\right]\sdot12=\left[\frac{1}{2}\sdot26\right]\sdot12=13\sdot12=156}}</math> | ||
|- | |- | ||
| − | |||
| | | | ||
| + | :If you want to extend upwards the two sides of the trapezoid, which are lines AB and GD, until they meet at point Z, as you can see in the shape, and this shape is like a triangle. | ||
| + | |style="text-align:right;"|ואם תרצה להאריך למעלה שני צלעי הקטומה שהם קו א"ב וג"ד עד שיפגשו אל נקודת ז' כמו שתראה בצורה ותהיה הצורה דומה למשולש | ||
|- | |- | ||
| | | | ||
| − | : | + | :[[File:Kelalim ba-Mispar 118-2.png|thumb|200px|left]] |
| − | : | + | |[[File:כללים במספר - קיח -2.png|thumb|200px]] |
| − | + | |- | |
| − | + | | | |
| − | |style="text-align:right;"| | + | :You want to know the length of the lines that go up, which are lines AZ and GZ. |
| − | + | |style="text-align:right;"|ותרצה לדעת אורך הקוים העולים למעלה והם קוי א"ז וג"ז | |
| − | + | |- | |
| − | |- | + | | |
| − | | | + | :Know the excess of the lower base over the upper base, which is 10 in this shape, and keep it. |
| − | : | + | |style="text-align:right;"|תדע עודף התושבת על הראש שהוא י' בצורה הזאת ותשמור אותו עמך |
| − | | | + | |- |
| − | |- | + | | |
| − | | | + | :[Multiply] the upper base, which is 8, by the whole side, which is 13; the total is 104. |
| − | :: | + | |style="text-align:right;"|ושוב ותראה הראש שהוא ח' בכל הצלע ‫<ref>112r</ref>שהוא י"ג ויהיה הכל ק"ד |
| − | | | + | |- |
| − | + | | | |
| − | + | :Divide it by 10, which is the excess you kept; the quotient is 10 and two-fifths and this is the length of line AZ, as well as line GZ, that go up to the point. | |
| − | + | |style="text-align:right;"|חלקם על י' אשר הוא העודף אשר שמרת ותהיה החלוקה י' ושני חומשי' והוא אורך קו א"ז וכמו כן קו ג"ז העולים עד הנקודה | |
| − | |style="text-align:right;"| | + | |- |
| − | |- | + | |colspan=2| |
| − | | | + | :<math>\scriptstyle{\color{blue}{AC=GC={\color{OliveGreen}{\frac{\left(\rm{bottom\ base}\right)\sdot\rm{side}}{\left(\rm{bottom\ base}\right)-\left(\rm{top\ base}\right)}}}=\frac{8\sdot13}{18-8}=\frac{104}{10}=10+\frac{2}{5}}}</math> |
| − | :{{ | + | |- |
| − | + | | | |
| − | + | :Or, do it this way: know the ratio of 8 cubits, which is the length of the upper base, to 10, which is the excess of the lower base over the upper base. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|או תעשה על זה הדרך שתדע ערך ח' אמות שהוא אורך הראש מי' שהוא עודף התושבת על הראש |
| − | + | |- | |
| − | + | | | |
| − | + | :The ratio of 8 to 10 is 4-fifths, i.e. 1 minus a fifth. | |
| − | + | |style="text-align:right;"|וערך ח' אל י' הוא ד' חמשי' דהיינו א' פחות חומש | |
| − | + | |- | |
| − | |style="text-align:right;"| | + | | |
| − | |- | + | :As the ratio of 8 to 10 so is the ratio of the line that goes up to point Z to 13, which is the side. |
| − | | | + | |style="text-align:right;"|וכערך ח' אל י' כן יהיה ערך הקו העולה עד נקודת הז' אל י"ג שהוא הצלע |
| − | : | + | |- |
| − | |style="text-align:right;"| | + | |colspan=2| |
| − | |- | + | :<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle AC:13=AC:BC=GC:13&\scriptstyle=GC:DC=AG:\left(BD-AG\right)\\&\scriptstyle={\color{OliveGreen}{\left(\rm{top\ base}\right):\left[\left(\rm{bottom\ base}\right)-\left(\rm{top\ base}\right)\right]}}\\&\scriptstyle=8:\left(18-8\right)=8:10=\frac{4}{5}=1-\frac{1}{5}\\\end{align}}}</math> |
| − | | | + | |- |
| − | : | + | | |
| − | + | :I.e. they are 13 minus 13-fifths, which is 10 and 2-fifths. | |
| − | + | :<math>\scriptstyle{\color{blue}{AC=GC=13-\frac{13}{5}=10+\frac{2}{5}}}</math> | |
| − | + | |style="text-align:right;"|דהיינו שיהיו י"ג פחות י"ג חומשי' שהם י' וב' חמשים וכה"ל | |
| − | + | |- | |
| − | : | + | | |
| − | + | ||
| − | + | ===== <span style=color:green>Trapezoid</span> ===== | |
| − | | | + | |
| − | + | | | |
| − | + | |- | |
| − | |- | + | | |
| − | | | + | :119) the area of this trapezoid is the product of one of its heights by half the sum of the upper base and the lower base together |
| − | ::<math>\scriptstyle{\color{ | + | :<math>\scriptstyle{\color{OliveGreen}{\rm{height}\sdot\left[\frac{1}{2}\sdot\left[\left(\rm{top\ base}\right)+\left(\rm{bottom\ base}\right)\right]\right]}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|קיט <big>{{#annot:term|816,1531|ez7p}}רבוע{{#annotend:ez7p}}</big> ''ה{{#annot:term|1094,2204|0OmP}}קטומה{{#annotend:0OmP}}'' הזאת הוא בריבוע אחד מעמודה במחצית הראש והתושבת יחד |
| − | + | |- | |
| − | |- | + | | |
| − | | | + | :[[File:Kelalim ba-Mispar 119.png|thumb|200px|left]] |
| − | : | + | |[[File:כללים במספר - קיט.png|thumb|200px]] |
| − | | | + | |- |
| − | + | | | |
| − | | | + | *{{#annot:trapezoid|680|ztT7}}In this shape, sum 8, which is the length of the upper base is 8, with 22, which is the lower base; the total sum is 30. |
| − | | | + | |style="text-align:right;"|והוא שתהיה מקבץ בצורה הזאת ח' שהוא אורך הראש כ"ב שהוא התושבת ויהיה הכל ל‫'{{#annotend:ztT7}} |
| − | |- | + | |- |
| − | | | + | | |
| − | + | :Take its half, which is 15, and multiply it by 12, which is the length of the height; the total is 180 and this is the area of this trapezoid. | |
| − | + | :<math>\scriptstyle{\color{blue}{12\sdot\left[\frac{1}{2}\sdot\left(8+22\right)\right]=12\sdot\left(\frac{1}{2}\sdot30\right)=12\sdot15=180}}</math> | |
| − | + | |style="text-align:right;"| קח מחציתו והוא ט"ו ומנה אותו בי"ב שהוא אורך העמוד ויהיה הכל ק"פ והוא יהיה תשבורת הקטומה הזאת וזאת היא הצורה | |
| − | + | |- | |
| − | :<math>\scriptstyle | + | | |
| − | |style="text-align:right;"| | + | |
| − | + | ===== <span style=color:green>Right Trapezoid</span> ===== | |
| − | + | ||
| − | + | | | |
| − | |- | + | |- |
| − | | | + | | |
| − | :: | + | :{{#annot:right trapezoid|680|qkJr}}120) The area of this trapezoid: |
| − | : | + | |style="text-align:right;"|קכ <big>רבוע</big> ''הקטומה'' הזאת{{#annotend:qkJr}} |
| − | | | + | |- |
| − | + | | | |
| + | :[[File:Kelalim ba-Mispar 120.png|thumb|200px|left]] | ||
| + | |[[File:כללים במספר - קכ.png|thumb|200px]] | ||
| + | |- | ||
| + | | | ||
| + | :By summing its upper base with its lower base, then multiplying it by the height and this is its area. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{height\sdot\left[\frac{1}{2}\sdot\left[\left(top\ base\right)+\left(bottom\ base\right)\right]\right]}}</math> | ||
| + | |style="text-align:right;"|הוא ב{{#annot:term|154,2205|Jbg2}}אסיפת{{#annotend:Jbg2}} ראשה אל תושבתה ותקח מחצית' וימנה בעמוד והוא יהיה תשברתה | ||
| + | |- | ||
| + | | | ||
| + | *In this shape, sum its upper base with its lower base; their total sum is 28. | ||
| + | |style="text-align:right;"|ואם תאסוף בצורה הזאת ראשה אל תושבתה יהיה כללם כ"ח | ||
| + | |- | ||
| + | | | ||
| + | :Take its half, which is 14, and multiply it by 9, which is the length of the height; it is 126 and this is the area of this trapezoid. | ||
| + | :<math>\scriptstyle{\color{blue}{9\sdot\left[\frac{1}{2}\sdot\left(8+20\right)\right]=9\sdot\left(\frac{1}{2}\sdot28\right)=9\sdot14=126}}</math> | ||
| + | |style="text-align:right;"|קח מחציתם שהוא י"ד ותרבה אותו בט' שהוא אורך העמוד יהיו קכ"ו והוא תשבורת הקטומה הזאת | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ===== <span style=color:green>Trapezoid</span> ===== | ||
| + | |||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :{{#annot:trapezoid|680|mOso}}121) The area of this trapezoid: | ||
| + | |style="text-align:right;"|קכא <big>תשבורת</big> ''הקטומה'' הזאת{{#annotend:mOso}} | ||
| + | |- | ||
| + | | | ||
| + | :[[File:Kelalim ba-Mispar 121.png|thumb|200px|left]] | ||
| + | |[[File:כללים במספר - קכא.png|thumb|200px]] | ||
| + | |- | ||
| + | | | ||
| + | :By multiplying the height by half the upper base and half the lower base. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\rm{height}\sdot\left[\frac{1}{2}\sdot\left[\left(\rm{top\ base}\right)+\left(\rm{bottom\ base}\right)\right]\right]}}</math> | ||
| + | |style="text-align:right;"|הוא ברבוע העמוד בחצי הראש וחצי התושבת | ||
| + | |- | ||
| + | | | ||
| + | *The sum of half the upper base and half the lower base is 17 and a half. | ||
| + | |style="text-align:right;"|וחצי הראש וחצי התושבת הם י"ז וחצי | ||
| + | |- | ||
| + | | | ||
| + | :Multiply it by 12, which is [the length of] the height; it is 210 and this is its area. | ||
| + | :<math>\scriptstyle{\color{blue}{12\sdot\left[\frac{1}{2}\sdot\left(14+21\right)\right]=12\sdot\left(17+\frac{1}{2}\right)=210}}</math> | ||
| + | |style="text-align:right;"|תרבה אותם על י"ב שהוא העמוד יהיו ר"י והוא תשברתו וכה"ל | ||
| + | |- | ||
| + | !<span style=color:green>Figure Problem - Quadrangle whose two sides are equal to its diagonal''' | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :{{#annot:quadrangle|680|v7pA}}122) Quadrangle whose two sides are equal to its diagonal. | ||
| + | |style="text-align:right;"|קכב <big>מרובע</big> שני קוויה כמרובע אלכסונה{{#annotend:v7pA}} | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | [[File:כללים במספר - קכב.png|thumb|350px|center]] | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | [[File:Kelalim ba-Mispar 122.png|thumb|250px|center]] | ||
| + | |- | ||
| + | | | ||
| + | :<span style=color:green>No verbal description is given</span> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== <span style=color:green>Arc</span> ==== | ||
| + | |||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | ===== <span style=color:green>Half a Circle; Arc</span> ===== | ||
| + | |||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :{{#annot:half a circle|680|K1G4}}123) A shape of half a circle - if you want to know the area of this shape: | ||
| + | |style="text-align:right;"|קכג ''<big>צורת</big> חצי עגול'' אם תרצה לדעת תשבורת הצורה הזאת{{#annotend:K1G4}} | ||
| + | |- | ||
| + | | | ||
| + | :Multiply half the chord, which is half the diameter of the circle, by half the perimeter, which is called an arc; then you will find the area. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\left(\frac{1}{2}\sdot2r\right)\sdot\left(\frac{1}{2}\sdot\rm{perimeter\ of\ half\ circle}\right)}}</math> | ||
| + | |style="text-align:right;"|תרבע חצי המיתר שהוא חצי קוטר העגול בחצי הקו העקום הנקרא קשת ותמצא התשבורת | ||
| + | |- | ||
| + | | | ||
| + | :If you want to know the measure of the arc, multiply half the chord, which is 4, by 3 and a seventh; they are 12 cubits and 4-sevenths; and this is the measure of the arc, i.e. the circumference of the semicircle. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(\frac{1}{2}\sdot2r\right)\sdot\left(3+\frac{1}{7}\right)=4\sdot\left(3+\frac{1}{7}\right)=12+\frac{4}{7}}}</math> | ||
| + | |style="text-align:right;"|ואם תרצה לדעת מדת הקשת תכפול חצי המיתר שהוא ד' ג' פעמ' ושביעי' פעם ‫<ref>112v</ref>ויהיו י"ב אמות וד' שבעיות וכן יהיה מדת הקשת ר"ל הקו הסובב עקמומית זה החצי העגול | ||
| + | |- | ||
| + | | | ||
| + | :[[File:Kelalim ba-Mispar 123.png|thumb|200px|left]] | ||
| + | |[[File:כללים במספר - קכג.png|thumb|200px]] | ||
| + | |- | ||
| + | | | ||
| + | :If you want to know the area of this semicircle, multiply half the chord by half the perimeter, as we have said, i.e. say: 4 times 6 and 2-sevenths; it is 25 and a seventh; and this is the area of the semicircle. | ||
| + | :<math>\scriptstyle{\color{blue}{4\sdot\left[\frac{1}{2}\sdot\left(12+\frac{4}{7}\right)\right]=4\sdot\left(6+\frac{2}{7}\right)=25+\frac{1}{7}}}</math> | ||
| + | |style="text-align:right;"|ואם תרצה לדעת שברי זה החצי העגול תרבע כאשר אמרנו חצי המיתר בחצי הקו הסובב דהיינו שתאמ' ד' פעמ' ו' וב' שבעיות יהיו כ"ה ושביעית וכן יהיה תשבורת חצי העגול וכה"ל | ||
| + | |- | ||
| + | | | ||
| + | :Or, do it this way: multiply the chord by itself; subtract from it the square of its seventh and half its seventh; then take half the remainder and this is the area of the semicircle. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\frac{1}{2}\sdot\left[\left(2r\right)^2-\left[\left[\frac{1}{7}+\left(\frac{1}{2}\sdot\frac{1}{7}\right)\right]\sdot\left(2r\right)^2\right]\right]}}</math> | ||
| + | |style="text-align:right;"|או תעשה על דרך זה תרבע את המיתר בעצמו ותוציא מזה המרובע שביעיתו וחצי שביעיתו וקח מחצית הנשאר והוא יהיה תשבורת חצי העגול | ||
| + | |- | ||
| + | | | ||
| + | :In this shape we drew: multiply the chord, which is 8, by itself; it is 64. | ||
| + | |style="text-align:right;"|ובצורה הזאת אשר עשינו תרבע המיתר שהוא ח' בעצמו יהיו ס"ד | ||
| + | |- | ||
| + | | | ||
| + | :Subtract from it its seventh and half its seventh, which is 13 cubits and 5-sevenths; 50 cubits and 2-sevenths of a cubit remain. | ||
| + | |style="text-align:right;"|הוצא ממנו שבעיתו וחצי שביעיתו שהם י"ג אמות וה' שבעיות ישארו נ' אמות וב' שביעי אמה | ||
| + | |- | ||
| + | | | ||
| + | :Take its half, which is 25 and a seventh; and this is the area of the semicircle. | ||
| + | |style="text-align:right;"|קח מחציתם שהם כ"ה ושביעית והוא יהיה תשבורת חצי העגול | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle\frac{1}{2}\sdot\left[8^2-\left[\left[\frac{1}{7}+\left(\frac{1}{2}\sdot\frac{1}{7}\right)\right]\sdot8^2\right]\right]&\scriptstyle=\frac{1}{2}\sdot\left[64-\left[\left[\frac{1}{7}+\left(\frac{1}{2}\sdot\frac{1}{7}\right)\right]\sdot64\right]\right]=\frac{1}{2}\sdot\left[64-\left(13+\frac{5}{7}\right)\right]\\&\scriptstyle=\frac{1}{2}\sdot\left(50+\frac{2}{7}\right)=25+\frac{1}{7}\\\end{align}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ===== <span style=color:green>Arc, smaller than half a circle</span> ===== | ||
| + | |||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :{{#annot:shape smaller than half a circle|680|fF3v}}124) An arc that is smaller than half a circle | ||
| + | :Example of an arc that is smaller than half a circle: arc ABG; its chord AG is 8 in length; its versed sine DB is 2 in length. | ||
| + | |style="text-align:right;"|קכד ''<big>קשת</big> פחות מחצי עגול''<br> | ||
| + | הדמיון לצורה המעוטה מחצי העגולה היא צורת קשת אב"ג ומיתרה א"ג ואורכו ח' וחיצה ד"ב ואורכו ב‫'{{#annotend:fF3v}} | ||
| + | |- | ||
| + | | | ||
| + | :[[File:Kelalim ba-Mispar 124-1.png|thumb|200px|left]] | ||
| + | |[[File:כללים במספר - קכד -1.png|thumb|200px]] | ||
| + | |- | ||
| + | | | ||
| + | :You cannot find the area of this shape if you do not know the whole diameter of the circle from which this arc is cut off. | ||
| + | |style="text-align:right;"|ואי אתה יכול להגיע לתשבורת הצורה הזאת אם אינך יודע כל קוטר העגול שזה הקשת נקטם ממנו | ||
| + | |- | ||
| + | | | ||
| + | :You can find the diameter of the circle this way: square half the chord; divide its square by the versed sine; then, add the measure of the versed sine to it; know the total and this is the length of the whole diameter. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{2r=\frac{\left(\frac{1}{2}\sdot\rm{chord}\right)^2}{\rm{versed\ sine}}+\rm{versed\ sine}}}</math> | ||
| + | |style="text-align:right;"|ותוכל לדעת קוטר העגול בעניין זה תרבע מחצית המיתר ותחלק מרובעו על החץ והוסף מדת החץ עליו ותדע סך הכל והוא יהיה אורך כל הקוטר | ||
| + | |- | ||
| + | | | ||
| + | :In the shape we drew: | ||
| + | |style="text-align:right;"|ומצורה הזאת אשר עשינו | ||
| + | |- | ||
| + | | | ||
| + | :Multiply half the chord, which is 4, by itself; it is 16. | ||
| + | |style="text-align:right;"|תרבע מחצית המיתר שהוא ד' בעצמו יהיו י"ו | ||
| + | |- | ||
| + | | | ||
| + | :Divide it by the versed sine, which is 2; it is 8 and this is the length of what remained from the diameter. | ||
| + | |style="text-align:right;"|וחלק אותו על החץ שהוא ב' יהיו ח' והוא האורך הנשאר מן הקוטר | ||
| + | |- | ||
| + | | | ||
| + | :Add 2 to it, which is the versed sine; it is 10. | ||
| + | |style="text-align:right;"|הוסף עליהם ב' שהוא החץ יהיו י‫' | ||
| + | |- | ||
| + | | | ||
| + | :So, the whole diameter of the circle from which this arc is cut off is 10. | ||
| + | :<math>\scriptstyle{\color{blue}{\frac{\left(\frac{1}{2}\sdot8\right)^2}{2}+2=\frac{4^2}{2}+2=\frac{16}{2}+2=8+2=10}}</math> | ||
| + | |style="text-align:right;"|הרי שכל הקוטר מן העגול שזה הקשת נקטם ממנו הוא י' וכה"ל | ||
| + | |- | ||
| + | | | ||
| + | :To clarify the matter well, complete the whole circle: | ||
| + | |style="text-align:right;"|ולראות העניין באר הטב הוי משלים העגולה הזאת כלה | ||
| + | |- | ||
| + | | | ||
| + | :Draw line BD that reaches the circumference of the circle on the other side, as line BDZ. | ||
| + | |style="text-align:right;"|והוצא קו ב"ד עד שיגיע אל עקמימות העגולה מן הצד השני כגון קו בד"ז | ||
| + | |- | ||
| + | | | ||
| + | :If you want to know the area of this shape: | ||
| + | |style="text-align:right;"|ואם תרצה לידע תשבורת הצורה הזאת | ||
| + | |- | ||
| + | | | ||
| + | :Divide line BZ into two equal parts at point C, which will be the center of the circle | ||
| + | |style="text-align:right;"|חלק קו ב"ז לב' חלקי' שוים על נקודת ח' ותהיה הנקודה הזאת {{#annot:term|1108,2178|0qCr}}ציר העגולה{{#annotend:0qCr}} | ||
| + | |- | ||
| + | | | ||
| + | :Draw from it two lines to point A and point G, which are lines CA and CG. | ||
| + | |style="text-align:right;"|ותרצה והוצא ממנה ב' קווים אל נקודת א' ואל נקודת ג' והם קוי ח"א וח"ג | ||
| + | |- | ||
| + | | | ||
| + | :[[File:Kelalim ba-Mispar 124-2.png|thumb|200px|left]] | ||
| + | |[[File:כללים במספר - קכד -2.png|thumb|200px]] | ||
| + | |- | ||
| + | | | ||
| + | :If you multiply line AC, which is half the diameter of the circle, by half the arc, which is arc AG, this product is the area of the triangle, whose two sides are the two lines AC and CG. | ||
| + | :<math>\scriptstyle{\color{blue}{\triangle_{ACG}=AC\sdot\left(\frac{1}{2}\sdot AG\right)}}</math> | ||
| + | |style="text-align:right;"|ואם אתה מרבע קו א"ח שהוא חצי הקוטר העגול בחצי הקשת אשר הוא עוקם א"ג יהיה מנין הזה תשבורת המשולש אשר ב' צלעיו הם ב' קוי א"ח וח"ג | ||
| + | |- | ||
| + | | | ||
| + | :Subtract the area of triangle ACG from the area of [sector] ABG and you are left with the area of [segment] ABGD. | ||
| + | |style="text-align:right;"|ותשבורת קשת אב"ג הוצא מן המנין תשבורת המשולש אח"ג וישאר בידך תשבורת הצורה שעליה אבג"ד | ||
| + | |- | ||
| + | | | ||
| + | :The area of this triangle is the product of line CD, which is 3 cubits in this shape, by half line AG, which is 4 cubits; their product is 12. | ||
| + | :<math>\scriptstyle{\color{blue}{ACG_{\triangle}=CD\sdot\left(\frac{1}{2}\sdot AG\right)=3\sdot4=12}}</math> | ||
| + | |style="text-align:right;"|ותשבורת המשולש הזה הוא רבוע קו ח"ד שהוא בצורה הזאת ג' אמות בחצי קו א"ג והוא ד' אמות ורבועם י"ב | ||
| + | |- | ||
| + | | | ||
| + | :Subtract this number from the product of the straight line AC by the arc AB; the remainder is the area of shape ABGD, which is the arc that is smaller than a semicircle. | ||
| + | |style="text-align:right;"|והוא הסך אשר תוציא מרבוע קו א"ח הישר בקו א"ב העקום ויהיה הנשאר תשבורת צורת אבג"ד שהוא צורת הקשת שהוא פחות מחצי עגול | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{ABGD=AG_{sector}-ACG_{\triangle}=AH\sdot\left(\frac{1}{2}\sdot AG_{arc}\right)-ACG_{\triangle}=\left[r\sdot\left(\frac{1}{2}\sdot AG_{arc}\right)\right]-ACG_{\triangle}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :Therefore, you say that the area of the arc that is smaller than a semicircle is [found] by that you multiply half the whole diameter of the circle, from which the arc is cut off, by half the arc; keep it. | ||
| + | |style="text-align:right;"|ומכאן אתה אומ' כי {{#annot:term|816,1531|9C3m}}רבוע{{#annotend:9C3m}} הקשת שהוא פחות מחצי עגול הוא שתרבע חצי כל הקוטר מן העגול שהקשת נקטם ממנו בחצי הקשת ותשמור אותו | ||
| + | |- | ||
| + | | | ||
| + | :Then, you subtract the versed sine of the arc by half the diameter and multiply what remains from half the diameter by half the chord. | ||
| + | |style="text-align:right;"|ואחרי כן תוציא מחצי הקוטר קו החץ אשר לקשת והנשאר מחצי הקוטר מנה אותו במחצית המיתר | ||
| + | |- | ||
| + | | | ||
| + | :You subtract the product from the number you kept and what you have left is the area of the arc that is less than a semicircle. | ||
| + | |style="text-align:right;"|והעולה בידך תפחות אותו מן המנין אשר שמרת בידך והנשאר בידך הוא תשבורת הקשת שהוא ‫<ref>113r</ref>פוחת מחצי עגול | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\left[\left[\frac{1}{2}\sdot\left(2r\right)\right]\sdot\left(\frac{1}{2}\sdot\rm{arc}\right)\right]-\left[\left[\left[\frac{1}{2}\sdot\left(2r\right)\right]-\rm{versed\ sine}\right]\sdot\left(\frac{1}{2}\sdot\rm{chord}\right)\right]}}</math> | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ===== <span style=color:green>Arc, greater than half a circle</span> ===== | ||
| + | |||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :{{#annot:shape greater than half a circle|680|DEQW}}125) The shape of the arc that is greater than half the circle, which is arc ABG; its chord AG is 12 in length. | ||
| + | |style="text-align:right;"|קכה <big>צורת</big> ''הקשת העודף על חצי העגול''<br> | ||
| + | והוא קשת אשר עליו אב"ג ומיתרו א"ג ואורכו י"ב{{#annotend:DEQW}} | ||
| + | |- | ||
| + | | | ||
| + | :[[File:Kelalim ba-Mispar 125.png|thumb|200px|left]] | ||
| + | |[[File:כללים במספר - קכה.png|thumb|200px]] | ||
| + | |- | ||
| + | | | ||
| + | :If you want to know the area of this shape, draw the diameter of the whole circle, from which this shape is cut off. Multiply half the diameter by half the arc, then add to it the area of the triangle, whose base is the chord. The total is the area of this shape. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\left[\left[\frac{1}{2}\sdot\left(2r\right)\right]\sdot\left(\frac{1}{2}\sdot\rm{arc}\right)\right]+\rm{Area}_{\triangle}}}</math> | ||
| + | |style="text-align:right;"|ואם תרצה לדעת תשבורת הצורה הזאת תוציא קוטר כל העגול שזאת הצורה נקטמת ממנו ותרבה חצי הקוטר בחצי הקשת ותוסיף עליו תשבורת המשולש אשר המיתר הוא תושבתו ויהיה סך הכל תשבורת כל זאת הצורה | ||
| + | |- | ||
| + | | | ||
| + | :In this shape, multiply half the chord, which is 6, by itself; the result is 36. | ||
| + | |style="text-align:right;"|ובצורה הזאת תרבע חצי המיתר שהוא ו' בעצמו שיבואו ל"ו | ||
| + | |- | ||
| + | | | ||
| + | :Divide it by the versed sine, which is 12; the quotient is 3. | ||
| + | |style="text-align:right;"|חלק אותם על החץ שהוא י"ב ויהיה החלוקה ג‫' | ||
| + | |- | ||
| + | | | ||
| + | :Add it to the versed sine; the total is 15 and this is the length of the whole diameter of the circle, from which this shape is cut off. | ||
| + | :<math>\scriptstyle{\color{blue}{\frac{\left(\frac{1}{2}\sdot12\right)^2}{12}+12=\frac{6^2}{12}+12=\frac{36}{12}+12=3+12=15}}</math> | ||
| + | |style="text-align:right;"|הוסיפם על החץ יהיה סך הכל ט"ו והוא אורך כל הקוטר מן העגול שזאת הצורה נקטמת ממנה | ||
| + | |- | ||
| + | | | ||
| + | :Its half is half the diameter and it is line BZ in this shape, so line BZ is 7 and a half. | ||
| + | :<math>\scriptstyle{\color{blue}{BZ=r=\frac{1}{2}\sdot15=7+\frac{1}{2}}}</math> | ||
| + | |style="text-align:right;"|ומחציתו הוא מחצית הקוטר והוא בצורה הזאת קו ב"ז הרי שקו ב"ז הוא ז' וחצי | ||
| + | |- | ||
| + | | | ||
| + | :Multiply it by half the arc; the result is the area of the segment encompassed by the two lines GZ and AZ and arc ABG. | ||
| + | :<math>\scriptstyle{\color{blue}{ABGZA=BZ\sdot\left(\frac{1}{2}\sdot\rm{arc}_{ABG}\right)}}</math> | ||
| + | |style="text-align:right;"|מנה אותו במחצית הקשת ומה שיעלה יהיה תשבורת הצורה העקומה אשר יקיפו אותה ב' קווי ג"ז וא"ז עם קשת א'ב'ג‫' | ||
| + | |- | ||
| + | | | ||
| + | :Add to it the area of triangle AZG, which is the product of line DZ by half line AG. When you sum all, it is the area of this segment. | ||
| + | |style="text-align:right;"|הוסף עליהם תשבורת משולש א'ז'ג' והוא המספר הנקבץ מרובע קו ד"ז במחצית קו א"ג וכשתקבץ הכל יהיה תשבורת העקמומית הזאת | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{ABGDA=ABGZA+AZG=ABGZA+\left[DZ\sdot\left(\frac{1}{2}\sdot AG\right)\right]}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :You learn from this that whoever wants to know the area of the segment that is greater than the semicircle, multiplies half the diameter by half the arc and keeps the number. | ||
| + | |style="text-align:right;"|ומכאן אתה למד הרוצה לדעת תשבורת העקום העודף על חצי העגול יהיה מרבע חצי הקוטר בחצי הקשת וישמור המספר | ||
| + | |- | ||
| + | | | ||
| + | :Then, subtracts half the diameter from the versed sine. | ||
| + | |style="text-align:right;"|ואחרי כן יפחות חצי הקוטר מן החץ | ||
| + | |- | ||
| + | | | ||
| + | :Multiplies what remains from the versed sine by half the chord. | ||
| + | |style="text-align:right;"|‫[והנשאר מן החץ] ירבע אותו בחצי המיתר | ||
| + | |- | ||
| + | | | ||
| + | :Adds the result to the number he kept and the total is the area of the segment that is greater than the semicircle. | ||
| + | |style="text-align:right;"|והמספר העולה יאספינו אל המספר אשר שמר ויהיה הכל סך תשבורת העקומה העודפת על חצי העגול | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\left[\left[\frac{1}{2}\sdot\left(2r\right)\right]\sdot\left(\frac{1}{2}\sdot\rm{arc}\right)\right]+\left[\left[\rm{versed\ sine}-\left[\frac{1}{2}\sdot\left(2r\right)\right]\right]\sdot\left(\frac{1}{2}\sdot\rm{chord}\right)\right]}}</math> | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | === <span style=color:green>Divide a Figure</span> === | ||
| + | |||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | ==== <span style=color:green>Triangle</span> ==== | ||
| + | |||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :{{#annot:triangle - two parts|686|cDS5}}126) If you want to divide the triangle into two equal parts [= the areas of both are equal] such that one part is a triangle and the other is a quadrangle | ||
| + | |style="text-align:right;"|קכו <big>אם</big> תרצה לחלק המשולש לב' חלקי' שוים וישאר החלק האחד משולש והשני דומה למרובע{{#annotend:cDS5}} | ||
| + | |- | ||
| + | | | ||
| + | *As triangle ABG, whose top is point A and its base is BG, and you want to divide it in half: | ||
| + | |style="text-align:right;"|כגון משולש א'ב'ג' אשר ראשו נקודת א' ותושבתו ב"ג ותרצה לחלקו לחצי | ||
| + | |- | ||
| + | | | ||
| + | :You should divide each of the sides AB and AG in two parts, so that the square of the whole side is twice the square of the larger portion of that side. | ||
| + | |style="text-align:right;"|אתה צריך לחלק כל אחד מצלעי א"ב וא"ג לב' חלקי' בענין שיהיה מרובע הצלע כולו כפליים ממרובע הצלע החלק הגדול מחלקיו | ||
| + | |- | ||
| + | | | ||
| + | :As if you divide line AB in two parts at point D and line AG in two parts at point H. | ||
| + | |style="text-align:right;"|כגון שתהיה חולק קו א"ב לב' חלקים על נקודת ד' וקו א"ג לב' חלקי' על נקודת ה‫' | ||
| + | |- | ||
| + | | | ||
| + | :[[File:Kelalim ba-Mispar 126.png|thumb|200px|left]] | ||
| + | |[[File:כללים במספר - קכו.png|thumb|200px]] | ||
| + | |- | ||
| + | | | ||
| + | :Let the square of AD be half the square of AB. | ||
| + | :<math>\scriptstyle{\color{blue}{AD^2=\frac{1}{2}\sdot AB^2}}</math> | ||
| + | |style="text-align:right;"|ויהיה מרובע א"ד מחצית מרובע א | ||
| + | |- | ||
| + | | | ||
| + | :Let also the square of AH be half the square of AG. | ||
| + | :<math>\scriptstyle{\color{blue}{AH^2=\frac{1}{2}\sdot AG^2}}</math> | ||
| + | |style="text-align:right;"|וכן מרובע א"ה מחצית מרובע א"ג | ||
| + | |- | ||
| + | | | ||
| + | :Draw a line from D to H, so that the triangle is divided into two equal parts: one part is triangle ADH and the other part is quadrilateral DHBG. | ||
| + | :<math>\scriptstyle{\color{blue}{\triangle_{ADH}=DHBG}}</math> | ||
| + | |style="text-align:right;"|ותוציא קו מד' על ה' והיה משולש נחלק לב' חלקי' שוים החלק האחד משולש א'ד'ה' וחלק השני נפתל ד'ה'ב'ג‫' | ||
| + | |- | ||
| + | | | ||
| + | :The way to calculate these squares is that you take 5 parts of 7 minus half a tenth of a seventh of the length of the line from point A, which is the top. The rule: you take 99 parts of 140 of the line. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\frac{5}{7}a-\left(\frac{1}{2}\sdot\frac{1}{10}\sdot\frac{1}{7}\right)a=\frac{99}{140}a}}</math> | ||
| + | |style="text-align:right;"|ודרך חשבון המרובעים האלה יהיה אם תהיה לוקח מאורך הקו מנקודת א' אשר הוא הראש ה' חלקי' מז' בו פחות חצי עשירית השביעית והכלל המסור לזה הוא שתהיה לוקח מן הקו צ"ט חלקי' מק"מ חלקי' בו | ||
| + | |- | ||
| + | | | ||
| + | *As if the length of side AB is 7 cubits. | ||
| + | |style="text-align:right;"|כאילו היה אורך צלע א"ב ז' אמות | ||
| + | |- | ||
| + | | | ||
| + | :Line AD is 5 cubits minus one part of twenty of a cubit. | ||
| + | :<math>\scriptstyle{\color{blue}{AD=5-\frac{1}{20}}}</math> | ||
| + | |style="text-align:right;"|יהיה קו א"ד מהם ה' אמות פחות חלק אחד מעשרים באמה | ||
| + | |- | ||
| + | | | ||
| + | :The length of side AG is 10 cubits. | ||
| + | |style="text-align:right;"|וצלע א"ג היה אורכו י' אמות | ||
| + | |- | ||
| + | | | ||
| + | :Line AH is 7 cubits and one part of 14 of a cubit. | ||
| + | :<math>\scriptstyle{\color{blue}{AH=7+\frac{1}{14}}}</math> | ||
| + | |style="text-align:right;"|יהיה קו א"ה ז' אמות וחלק אחד מי"ד באמה | ||
| + | |- | ||
| + | | | ||
| + | :Divide both sides according to this calculation, and draw a line from one part to the other, then the triangle is divided into two equal parts. | ||
| + | |style="text-align:right;"|ועל החשבון הזה אתה חולק את שתי הצלעות האלה ותוציא קו מן החלק האחד אל השני ויחלק המשולש לב' חלקים שוים | ||
| + | |- | ||
| + | | | ||
| + | ==== <span style=color:green>Triangular Field</span> ==== | ||
| + | |||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :{{#annot:triangular field - three owners|686|a7VB}}127) If you have to divide a triangular field to three owners and each asks for his share in accordance with one of the sides of the triangle | ||
| + | |style="text-align:right;"|‫<ref>113v</ref>קכז <big>ואם</big> יהיה קרקע המשולש לג' בעלים ואתה צריך לחלקו ביניהם וכל אחד מהבעלים מבקש חלקו נגד צלע אחד מצלעי המשולש{{#annotend:a7VB}} | ||
| + | |- | ||
| + | | | ||
| + | *Let the triangle be triangle ABG. | ||
| + | |style="text-align:right;"|ויהיה המשולש הזה משולש א'ב'ג‫' | ||
| + | |- | ||
| + | | | ||
| + | :You divide side AB into two equal parts at point D. | ||
| + | :<math>{\color{blue}{\scriptstyle AD=BD}}</math> | ||
| + | |style="text-align:right;"|ואתה בא וחלק צלע א"ב לב' חלקי' שוים על נקודת ד‫' | ||
| + | |- | ||
| + | | | ||
| + | :Draw a line from D to G, so the triangle is divided into two equal parts. | ||
| + | |style="text-align:right;"|והוצא קו מד' אל ג' ויחלק המשולש לב' חלקי' שוי‫' | ||
| + | |- | ||
| + | | | ||
| + | :[[File:Kelalim ba-Mispar 127.png|thumb|200px|left]] | ||
| + | |[[File:כללים במספר - קכז.png|thumb|200px]] | ||
| + | |- | ||
| + | | | ||
| + | :Then, measure a third of line DG, which is the height if the triangle, from point D that is on the base. Let this part be line DH. | ||
| + | :<math>{\color{blue}{\scriptstyle DH=\frac{1}{3}\sdot DG}}</math> | ||
| + | |style="text-align:right;"|ואחר כן מדוד מקו ד"ג אשר הוא קומת המשולש שלישיתו מנגד נקודת ד' אשר על התושבת ויהיה החלק הזה קו ד"ה | ||
| + | |- | ||
| + | | | ||
| + | :Draw from point H two lines to point A and to point B. | ||
| + | |style="text-align:right;"|והוצא מנקודת ה' ב' קוים אל נקודת א' ואל נקודת ב‫' | ||
| + | |- | ||
| + | | | ||
| + | :The triangle is divided into three equal parts: triangle GHA, triangle AHB, and triangle GHB. | ||
| + | :<math>\scriptstyle{\color{blue}{\triangle GHA=\triangle AHB=\triangle GHB}}</math> | ||
| + | |style="text-align:right;"|ויחלק המשולש לג' חלקים שוים והם משולש ג'ה'א' ומשולש א'ה'ב' ומשולש ג'ה'ב‫' | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | === <span style=color:green>Rhombus</span> === | ||
| + | |||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :{{#annot:rhombus|679|n38W}}128) Questions concerning the rhombus mentioned above - one of its diagonals is 16 and the other is 12. | ||
| + | :How much is the side? | ||
| + | |style="text-align:right;"|קכח <big>שאלות</big> במעויין הנז' למעלה אשר אחד מאלכסוניו י"ו והשני י"ב<br> | ||
| + | כמה הוא צלעו{{#annotend:n38W}} | ||
| + | |- | ||
| + | | | ||
| + | :Answer: take half of each of its diagonals, square them, sum the two squares and extract the root [of their sum]; then you will find the side. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\sqrt{\left(\frac{1}{2}\sdot\rm{diagonal}_1\right)^2+\left(\frac{1}{2}\sdot\rm{diagonal}_2\right)^2}}}</math> | ||
| + | |style="text-align:right;"|תשובה קח מחצית כל אחד מאלכסוניו ורבע אותם וקבץ שני המרובעי' וקח את גדרם ותמצא הצלע | ||
| + | |- | ||
| + | | | ||
| + | :Explanation of the answer: | ||
| + | |style="text-align:right;"|ופירוש התשובה | ||
| + | |- | ||
| + | | | ||
| + | :Take the half of 16; it is 8 and its square is 64. | ||
| + | |style="text-align:right;"|קח מחצית י"ו והוא ח' וריבועו ס"ד | ||
| + | |- | ||
| + | | | ||
| + | :Take the half of 12; it is 6 and its square is 36. | ||
| + | |style="text-align:right;"|וקח מחצית י"ב והוא ו' וריבועו ל"ו | ||
| + | |- | ||
| + | | | ||
| + | :The sum of these two squares is 100. | ||
| + | |style="text-align:right;"|ומניין שני המרובעים האלו הם ק‫' | ||
| + | |- | ||
| + | | | ||
| + | :The root of 100 is 10 and this is the side of the rhombus. | ||
| + | |style="text-align:right;"|וגדר ק' הם י' והוא צלע המרובע | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{\left(\frac{1}{2}\sdot16\right)^2+\left(\frac{1}{2}\sdot12\right)^2}=\sqrt{8^2+6^2}=\sqrt{64+36}=\sqrt{100}=10}}</math> | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:rhombus|683|EFrZ}}If it is said: the area of the rhombus is 96, and one of its diagonals is 16. | ||
| + | :How much is the other diagonal? | ||
| + | |style="text-align:right;"|ואם יאמר {{#annot:term|1095,1526|gBop}}מעויין{{#annotend:gBop}} שתשברתו צ"ו ואלכסון האחד י"ו<br> | ||
| + | כמה הוא האלכסון השני{{#annotend:EFrZ}} | ||
| + | |- | ||
| + | | | ||
| + | :Divide 96, which is the area, by the diagonal that is known to be 16; you find that half the other diagonal is 6; its double is 12 and this is the other diagonal. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\rm{diagonal}_2=2\sdot\frac{\rm{area_{rhombus}}}{diagonal_1}}}{\color{blue}{=2\sdot\frac{96}{16}=2\sdot6=12}}</math> | ||
| + | |style="text-align:right;"|חלק צ"ו אשר הוא התשבורת על האלכסון אשר ידעת שהוא י"ו ותמצא מחצית האלכסון השני ו' וכפלה י"ב והוא האלכסון השני | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:rhombus|683|R9Pd}}If it is said: the side of the rhombus is 10 and its area is 96. | ||
| + | :How much are its diagonals? | ||
| + | |style="text-align:right;"|ואם יאמ' מעויין צלעו י' ותשברתו צ"ו<br> | ||
| + | כמה אלכסונו{{#annotend:R9Pd}} | ||
| + | |- | ||
| + | | | ||
| + | :Take the square of the side, which is 100. Add the area to it; it is 196. The root of this number is 14 and this is [the sum of] the two halves of the diagonals. | ||
| + | |style="text-align:right;"|קח מרובע הצלע והוא ק' הוסף עליו התשבורת ויהיו קצ"ו וגדר המספר הזה הוא י"ד והם שני חציי האלכסונות | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\frac{1}{2}\sdot\rm{diagonal}_1+\frac{1}{2}\sdot\rm{diagonal}_2=\sqrt{\rm{side^2+area}}}}{\color{blue}{=\sqrt{10^2+96}=\sqrt{100+96}=\sqrt{196}=14}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :Take halve the number, which is 7. Its square is 49. | ||
| + | |style="text-align:right;"|קח מחצית המספר והוא ז' ומרובעו מ"ט | ||
| + | |- | ||
| + | | | ||
| + | :Subtract from it half the area, which is 48; you are left with 1. | ||
| + | |style="text-align:right;"|הוצא ממנו מחצית התשבורת אשר הוא מ"ח וישאר בידך א‫' | ||
| + | |- | ||
| + | | | ||
| + | :The root of 1 is 1. If you add it to 7, they are 8 and this is a half of the one diagonal. | ||
| + | |style="text-align:right;"|וגדר הא' הוא א' אם אתה מוסיף אותו על ז' יהיו ח' והוא מחצית האלכסון האחד | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle\frac{1}{2}\sdot\rm{diagonal}_1&\scriptstyle=\left(\frac{1}{2}\sdot14\right)+\sqrt{\left(\frac{1}{2}\sdot14\right)^2-\left(\frac{1}{2}\sdot\rm{area}\right)}=7+\sqrt{7^2-\left(\frac{1}{2}\sdot96\right)}=7+\sqrt{49-48}\\&\scriptstyle=7+\sqrt{1}=7+1=8\\\end{align}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :If you subtract it from 7, six remains and this is a half of the second diagonal. | ||
| + | |style="text-align:right;"|ואם אתה פוחת אותו מן ז' ישאר ששה והוא מחצית האלכסון השני | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{2}\sdot\rm{diagonal}_2=\left(\frac{1}{2}\sdot14\right)-\sqrt{\left(\frac{1}{2}\sdot14\right)^2-\left(\frac{1}{2}\sdot\rm{area}\right)}=7-1=6}}</math> | ||
| + | |- | ||
| + | !<span style=color:green>Figure Problem - Divide a Figure - Triangle</span> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :{{#annot:triangle - two parts|686|80ZX}}129) Question: a triangle, its one side AB is 12 cubits; the second side BG is 10 cubits and the third side AG is 15 cubits. | ||
| + | :You want to divide it to two owners equally. | ||
| + | |style="text-align:right;"|קכט <big>שאילה</big> משולש אשר צלעו האחד י"ב אמות והוא צלע א"ב וצלע ב"ג השני י' אמות וצלע א"ג השלישי ט"ו אמות<br> | ||
| + | ואתה רוצה לחלקו לב' בעלים לכל אחד חלק שוה{{#annotend:80ZX}} | ||
| + | |- | ||
| + | | | ||
| + | :[[File:Kelalim ba-Mispar 129.png|thumb|200px|left]] | ||
| + | |[[File:כללים במספר - קכט.png|thumb|200px]] | ||
| + | |- | ||
| + | | | ||
| + | :We mark point D two cubits away from point B on side AB. | ||
| + | :<math>\scriptstyle{\color{blue}{D_1B=2}}</math> | ||
| + | |style="text-align:right;"|והנה נשים נקודות ד' אשר הוא המיתר רחוקה מנקודת ב' על צלע א"ב שתי אמות | ||
| + | |- | ||
| + | | | ||
| + | :Line AD remains 10 cubits long. | ||
| + | :<math>\scriptstyle{\color{blue}{AD_1=AB-D_1B=10}}</math> | ||
| + | |style="text-align:right;"|וישאר קו א"ד י' אמות | ||
| + | |- | ||
| + | | | ||
| + | :Half line AB [is marked by] point Z. So, line AZ is six cubits, because we said that the whole side AB is 12 cubits long. | ||
| + | :<math>\scriptstyle{\color{blue}{AZ=BZ=\frac{1}{2}\sdot AB=6}}</math> | ||
| + | |style="text-align:right;"|ויהיה מחצית צלע א"ב על נקודת ז' ויהיה קו א"ז שש אמות כי כן מסרנו צלע א"ב כולו י"ב אמות | ||
| + | |- | ||
| + | | | ||
| + | :Between line AZ, which is the half [of AB], and line AD there are four cubits and they are two-fifths of line AD. | ||
| + | :<math>\scriptstyle{\color{blue}{D_1Z=AZ-AD_1=4=\frac{2}{5}\sdot AD_1}}</math> | ||
| + | |style="text-align:right;"|ובין קו א"ז שהוא המחצית ובין קו א"ד ארבע אמות<br> | ||
| + | והם שני חמישיות קו א"ד | ||
| + | |- | ||
| + | | | ||
| + | :Mark point H two-fifths from the top of line AG that is next to AD. | ||
| + | |style="text-align:right;"|ואתה קח מצלע א"ג הנדבק אל א"ד שני חמשיותיו אשר היא הראש על נקודת ה‫' | ||
| + | |- | ||
| + | | | ||
| + | :So, line GH is six cubits, because the whole side AG is 15 cubits. | ||
| + | :<math>\scriptstyle{\color{blue}{GH_1=\frac{2}{5}\sdot AG=\frac{2}{5}\sdot15=6}}</math> | ||
| + | |style="text-align:right;"|ויהיה קו ג"ה שש אמות כי כל צלע א"ג היה ט"ו אמה | ||
| + | |- | ||
| + | | | ||
| + | :Mark the half [by drawing a line] from point D to point H. | ||
| + | |style="text-align:right;"|והוצא המחצה מנקודת ד' עד נקודת ה‫' | ||
| + | |- | ||
| + | | | ||
| + | :The triangle is divided into two equal parts: one part is triangle DAH and the other part is quadrilateral DHGB. | ||
| + | :<math>\scriptstyle{\color{blue}{\triangle D_1AH_1=D_1H_1GB}}</math> | ||
| + | |style="text-align:right;"|ויהיה המשולש הזה נחלק לשני חלקי' שוים החלק האחד משולש ד'א'ה' והחלק השני נפתל ד"ה ג"ב | ||
| + | |- | ||
| + | | | ||
| + | :If point D [is closer to the other side] so that AD is two cubits, you should find the half of side BG instead of [the half of] side AG. | ||
| + | :<math>\scriptstyle{\color{blue}{AD_2=2}}</math> | ||
| + | |style="text-align:right;"|ואילו היה המיצר אשר הוא נקודת ד' נופל לצד אחד עד שיהיה א"ד שתי אמות לא היית מוצא המחצה אל הצלע א"ג אבל אל צלע ב"ג | ||
| + | |- | ||
| + | | | ||
| + | :Mark point H two-fifths from point G on line BG, which is 10 cubits. | ||
| + | :<math>\scriptstyle{\color{blue}{GH_2=\frac{2}{5}\sdot BG=\frac{2}{5}\sdot10=4}}</math> | ||
| + | |style="text-align:right;"|והיית לוקח מצלע ב"ג אשר הוא י' אמות שני חמשיותיו על נקודת ה' מנגד נקודת ג‫' | ||
| + | |- | ||
| + | | | ||
| + | :So, line GH is 4 cubits on side BG. | ||
| + | |style="text-align:right;"|ויהיה קו ג"ה ‫<ref>114r</ref>בצלע ב"ג ד' אמות | ||
| + | |- | ||
| + | | | ||
| + | :Mark the half [by drawing a line] from point D to point H as you see in this shape. | ||
| + | :<math>\scriptstyle{\color{blue}{\triangle D_2BH_2=D_2H_2GA}}</math> | ||
| + | |style="text-align:right;"|ותוציא המחצה מנקודת ד' אל נקודת ה' כאשר אתה רואה בצורה הזאת | ||
| + | |- | ||
| + | | | ||
| + | :Because the [crossing line] in this example is always drawn from the longer part: | ||
| + | |style="text-align:right;"|כי המחצה בדמיון הזה היא יוצאה לעולם אל החלק הארוך | ||
| + | |- | ||
| + | | | ||
| + | :If the longer [part] is BD - you draw the [crossing line] to side BG. | ||
| + | |style="text-align:right;"|אם יהיה הארוך ב"ד אתה מוציא המחצה אל צלע ב"ג | ||
| + | |- | ||
| + | | | ||
| + | :If the longer [part] is AD - you draw the [crossing line] to side AG. | ||
| + | |style="text-align:right;"|ואם יהיה הארוך א"ד אתה מוציא המחצה אל צלע א"ג | ||
| + | |- | ||
| + | | | ||
| + | :You start measuring the two-fifths from point G, which is the top vertex, and the [crossing line] is drawn from that point [to a point that is as far from the midpoint of side AB as that point is] from point G, which is the top vertex. | ||
| + | |style="text-align:right;"|ואתה מתחיל למדוד את שתי החמשיות מנקודת ג' אשר היא הראש ואל הנקודה הזאת הייתה המחצה יוצאה אלו היה המיצר נופל על מחצית צלע א"ב ומדת מרחק המיצר מן המחצית אתה מתרחק מנקודת ג' אשר הוא הראש | ||
| + | |- | ||
| + | | | ||
| + | :You can understand this issue without the numerical illustration, but I mentioned the numbers in order to make it easier for the student and bring the matter closer to the heart of the teacher. | ||
| + | |style="text-align:right;"|ואתה יכול להבין את העניין הזה אם לא היית מדמה אותו במספר<br> | ||
| + | אלא שזכרתי המספר כדי להקל על הלומד ולהקריב העניין ללב המלמד | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | === <span style=color:green>Area</span> === | ||
| + | |||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | ==== <span style=color:green>Polygons</span> ==== | ||
| + | |||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :{{#annot:polygon|680|TQAX}}130) Question in the measurement of shapes whose sides are more the four sides [= polygons]. These shapes are numerous, some of them are pentagonal, some of them are hexagonal and octagonal and so on, some of them have equal sides and angles and some are scalene. | ||
| + | |style="text-align:right;"|קל <big>שאלה</big> במדידת הצורות אשר צלעותיו עודפות על ארבע הצלעות והצורות האלה הם על תמונות רבות מהם מחומשות ומהם משותתות ומשובעות ולמעלה מהם יש מהם שצלעותם וזוויותם שוות ויש שלא יהיו שוות אלא מתחלפות{{#annotend:TQAX}} | ||
| + | |- | ||
| + | | | ||
| + | :First, I give you a rule for the measurement of equilateral polygons: | ||
| + | |style="text-align:right;"|ואני נותן לך כלל ראשונה במדידת הצורות אשר צלעותן וזוויותן שוות | ||
| + | |- | ||
| + | | | ||
| + | :This rule is that for every shape that has straight lines, if you circle a circle inside it that touches its sides, it is known that the product of half the diameter of this circle by half of all the sides of that shape is its area. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\left(\frac{1}{2}\sdot\rm{diameter_{incircle}}\right)\sdot\frac{1}{2}\sdot\sum \rm{sides}}}</math> | ||
| + | |style="text-align:right;"|והכלל הזה הוא כל תבנית וכל צורה אשר אין לה קוים ישרים אם אתה מעגיל בתוכה עגול שיהיה מושש לצלעיה ידוע הוא כי רבוע קוטר חצי העגול הזה במחצית כל צלעי הצורה ההיא א"ו היא תשברתה | ||
| + | |- | ||
| + | | | ||
| + | :For every [polygon] from the square onward whose sides and angles are equal to each other, you can draw a circle in it that touches all its sides. | ||
| + | |style="text-align:right;"|וכל צורה מן המרבע ולמעלה אשר צלעיה וזוויותיה שוות זו לזו אתה יכול {{#annot:term|2498,2218|Wkts}}לחוק בה עגול{{#annotend:Wkts}} שיהיה מושש לכל צלעיה | ||
| + | |- | ||
| + | | | ||
| + | :If their sides and angles are not equal to each other, for some of [these shapes] it is impossible to draw a circle in them that touches all their sides. | ||
| + | |style="text-align:right;"|ואם אין צלעיה וזוויותיה שוות יש מהם שלא יתכן {{#annot:term|2498,2218|CyVl}}לחוק בעגול{{#annotend:CyVl}} שיהיה מושש לכל צלעיה | ||
| + | |- | ||
| + | | | ||
| + | :If you have an [equilateral] triangle, square, pentagon or onward and you draw a circle inside it that touches its sides, know that the product of half the diameter of that circle by half [the sum] of the sides of this shape is its area. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\left(\frac{1}{2}\sdot\rm{diameter_{incircle}}\right)\sdot\frac{1}{2}\sdot\sum \rm{sides}}}</math> | ||
| + | |style="text-align:right;"|ואם תבא לידך צורה מכל התמונות מן משולש או מרובע או מחומש או למעלה מהם ואתה מחיק בתוכה עגולה מוששת לצלעיה<br> | ||
| + | הוי יודע כי רבוע חצי קוטר העגול ההוא במחצית כל צלעי התמונה הוא תשברתה | ||
| + | |- | ||
| + | | | ||
| + | :We do not need to give an example for the triangle and the square, because the way [to find] their area has already been explained. | ||
| + | |style="text-align:right;"|ואין אנו צריכין לתת דמיון מן המשולש ולא מן המרובע כי כבר פורש דרך רבועם | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:pentagon|680|jZu4}}However, we give an example for [finding the area of] the pentagon whose five angles are A, B, G, D, H, all its sides are equal in length and all its angles are equal to each other. | ||
| + | |style="text-align:right;"|אבל אנו נותנין דמיון מן המחומש והוא אשר על חמש זוויותיו א'ב'ג'ד'ה' וכל צלע וצלע מצלעותיו שוות באורכן וכן זוויותיו כלם שוות זו לזו{{#annotend:jZu4}} | ||
| + | |- | ||
| + | | | ||
| + | :We draw a circle in it that touches its five sides at five points Z, C, T, K, L. | ||
| + | |style="text-align:right;"|ואנו מעגילים בתוכן עגול מושש לה' צלעותיו על חמש נקודות ז'ח'ט'כ'ל‫' | ||
| + | |- | ||
| + | | | ||
| + | :The center of the circle is point M. | ||
| + | |style="text-align:right;"|ו{{#annot:term|1108,2178|a6OG}}ציר העגול{{#annotend:a6OG}} הזה הנקרא מרכז הוא נקודת מ‫' | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | [[File:כללים במספר - קל.png|thumb|300px|center]] | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | [[File:Kelalim ba-Mispar 130.png|thumb|300px|center]] | ||
| + | |- | ||
| + | | | ||
| + | :We say that the product of half the diameter of that circle by half [the sum] of all the sides of the pentagon is its area. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\left(\frac{1}{2}\sdot\rm{diameter_{incircle}}\right)\sdot\frac{1}{2}\sdot\sum_{i=1}^5 \rm{side}_i}}</math> | ||
| + | |style="text-align:right;"|ואנו אומרים כי רבוע חצי קוטר העגול הזה במחצית כל צלעי המחומש הוא תשברתו | ||
| + | |- | ||
| + | | | ||
| + | :Half the diameter is any line that goes from point M to one of the points where the circle touches the pentagon. | ||
| + | |style="text-align:right;"|וחצי הקוטר הוא קו יוצא מנקודת מ' אל אחת הנקודות אשר העגול מושש עליהם את המחומש | ||
| + | |- | ||
| + | | | ||
| + | :Now, if we draw a line from point M, which is the center, to the two points A and B that are vertices of the pentagon, it gives us triangle MAB. | ||
| + | |style="text-align:right;"|ועתה אם אנו מוצאים קו מנקודת מ' אשר הוא המרכז על שתי נקודות א' וב' מן זווית המחומש נתן לנו משולש אשר עליו מ'א'ב‫' | ||
| + | |- | ||
| + | | | ||
| + | :The area of this triangle is the product of the height drawn to its base by half the base. | ||
| + | |style="text-align:right;"|ותשבורת המשולש הזה הוא ברבוע העמוד היוצא אל תושבתו בחצי התושבת | ||
| + | |- | ||
| + | | | ||
| + | :If we draw a line from point M to point L, where the circle touches side AB, this line is the height of triangle MAB, which is half the diameter of the circle. | ||
| + | |style="text-align:right;"|ואם אנו מוציאים קו מנקודת מ' אל נקודת ל' אשר משש עליה העגול את צלע א"ב יהיה הקו הזה עמוד במשולש מ'א'ב' והוא חצי קוטר העגול | ||
| + | |- | ||
| + | | | ||
| + | :If we multiply this height by half the side AB, the product is the area of the triangle. | ||
| + | |style="text-align:right;"|ואם מרבע העמוד הזה בחצי צלע א"ב יהיה רבועו תשבורת המשולש | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | :<math>\scriptstyle{\color{blue}{\rm{height}\sdot\left(\frac{1}{2}\sdot AB\right)=ML\sdot\left(\frac{1}{2}\sdot AB\right)=\frac{1}{2}\sdot\rm{diameter}\sdot\left(\frac{1}{2}\sdot AB\right)}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :This way you can construct five triangles on the five sides of the pentagon, the area of each triangle is the product of half the diameter by half the side, as [illustrated] for side [AB], and [the sum of] the area of the five triangles is the area of the pentagon, so it is a product of half the diameter by [the sum of] half the five sides. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\left(\frac{1}{2}\sdot diameter_{incircle}\right)\sdot\frac{1}{2}\sdot\sum_{i=1}^5 side_i}}</math> | ||
| + | |style="text-align:right;"|ועל הדרך הזה אתה יכול לעשות חמשה משולשים על ‫<ref>114v</ref>חמש צלעי המשולש המחומש ויהיה תשבורת כל משולש מהם ברבוע חצי הקוטר בחצי הצלע כאשר היה בצלע הזה ותשבורת חמש המשולשים כולם הוא תשבורת המחומש והוא מרבוע חצי הקוטר בחצי כל חמש הצלעות | ||
| + | |- | ||
| + | | | ||
| + | :This is the case for any similar shape, which has many or few sides: if you draw a circle inside it that touches its sides, its area is the product of half the diameter of the circle by [the sum of] half of the sides. | ||
| + | |style="text-align:right;"|והוא יהיה העניין לכל צורה כמוה אשר צלעותיה רבים או מעטים אם אתה מעגיל בתוכה עגול מושש לצלעיה יהיה תשברתה ברבוע חצי קוטר העגול במחצית הצלעות וזאת היא צורתה | ||
| + | |- | ||
| + | | | ||
| + | :Since you cannot draw in every shape a circle that touches all its sides, this rule is not enough for you in measuring the shapes that have more than four sides. | ||
| + | |style="text-align:right;"|ומפני שאין אתה יכול להוציא בכל תמונה ותמונה עגול שיהיה מושש לכל צלעיה אין הכלל הזה מספיק לך במדידת כל העודפות על ד' צלעות | ||
| + | |- | ||
| + | | | ||
| + | :Therefore, I give you another rule that you can apply for any shape whose sides are straight [= polygons] [no proof is given]: | ||
| + | |style="text-align:right;"|ומפני זה אני נותן לך כלל אחר שיהיה נוהג לך בכל צורה אשר צלעותיה ישרות | ||
| + | |- | ||
| + | | | ||
| + | :Know that any plane shape whose sides are straight is divided into triangles [whose number] is less than the number of sides by two. | ||
| + | |style="text-align:right;"|הוי יודע כי כל תמונה שטוחה שצלעותיה ישרות מתחלקת למשולשים שהם חסרים מן מניין הצלעות שנים | ||
| + | |- | ||
| + | | | ||
| + | :As the square that has four sides is divided into two triangles, whose number is less than the number of sides by two. | ||
| + | |style="text-align:right;"|כגון המרובע אשר לו ד' צלעות יתחלק לב' משולשים אשר מניינם פוחת ב' ממספר הצלעות | ||
| + | |- | ||
| + | | | ||
| + | :The hexagon is divided into four triangles. | ||
| + | |style="text-align:right;"|והמשושה יתחלק לד' משולשים | ||
| + | |- | ||
| + | | | ||
| + | :And so on. | ||
| + | |style="text-align:right;"|וכן למעלה מזה | ||
| + | |- | ||
| + | | | ||
| + | :According to this rule, divide any shape you have into the triangles into which it is divided, and find the area of each triangle according to the way you have learned; [the sum of] all their areas is the area of the shape from which they were divided. | ||
| + | |style="text-align:right;"|ואתה בא מן הכלל הזה וחלק כל תמונה שיבוא לידך אל המשולשים אשר היא נחלקת אליהם ורבע כל משולש מהם על הדרך אשר למדת ויהיה מרובע כולם תשבורת התמונה אשר נחלקו ממנה | ||
| + | |- | ||
| + | | | ||
| + | *As if you draw a line from point A to point G and another line to point D in the pentagon that I have drawn for you above, so the pentagon is divided into three triangles. | ||
| + | |style="text-align:right;"|כאלו היית מוצא במחומש אשר ציירתי לך למעלה קו מנקודת א' אל נקודת ג' וקו אחר אל נקודת ד' ויהיה המחומש נחלק לג' משולשים | ||
| + | |- | ||
| + | | | ||
| + | :You cannot reduce [the number of] these triangles. | ||
| + | |style="text-align:right;"|ואין אתה יכול לפחות מהמשולשים האלה | ||
| + | |- | ||
| + | | | ||
| + | :If you find their areas, [the sum of] the areas of the three is the area of the pentagon. | ||
| + | |style="text-align:right;"|ואם אתה מרבע אותם יהיה רבוע שלשתם תשבורת המחומש כגון הצורה הזאת | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== <span style=color:green>Square</span> ==== | ||
| + | |||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :{{#annot:square|680|5Xvk}}131) You have a square of 10 cubits by 10 cubits within it a smaller square of 4 cubits by 4 cubits is drawn. | ||
| + | :You want to know how much is the area of the larger square after subtracting the area of the smaller square from it. | ||
| + | |style="text-align:right;"|קלא <big>הרי</big> שיש לפניך א' מרובע מי' אמה על י' אמה ובאמצע הוא חקוק ופוחת ד' אמות על ד' אמות<br> | ||
| + | ותרצה לדעת כמה הוא המרובע בשיבור אחר הוצאת הד' אמות על ד' אמות מן החקק{{#annotend:5Xvk}} | ||
| + | |- | ||
| + | | | ||
| + | :[[File:Kelalim ba-Mispar 131.png|thumb|200px|left]] | ||
| + | |[[File:כללים במספר - קלא.png|thumb|200px]] | ||
| + | |- | ||
| + | | | ||
| + | :Say: if the square were complete, you would say 10 times 10 is 100 and its area were 100. | ||
| + | |style="text-align:right;"|תאמר אלו היה הטבלה מרובעת שלימה בלתי שום חקיקה היית אומר י' פעמ' י' הם ק' ותשברתו היה ק‫' | ||
| + | |- | ||
| + | | | ||
| + | :Now, that 4 times 4 is missing in the middle, say 4 times 4 is 16. | ||
| + | |style="text-align:right;"|ועתה שפוחת באמצע ד' על ד' תאמ' ד' פעמ' ד' הם י"ו | ||
| + | |- | ||
| + | | | ||
| + | :Subtract it from 100; 84 remains. | ||
| + | |style="text-align:right;"|תפחתם מק' ישארו פ"ד | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | :<math>\scriptstyle{\color{blue}{\rm{area_{big\ square}}-\rm{area_{small\ square}}=10^2-4^2=100-16=84}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :So, the area of a square of 10 by 10 that is missing 4 by 4 in the middle is 84. | ||
| + | |style="text-align:right;"|א"כ א' טבלה מרובעת שהיא י' על י' ופוחת באמצעה ד' על ד' תשברתה פ"ד | ||
| + | |- | ||
| + | | | ||
| + | :The same for everything similar to it. | ||
| + | |style="text-align:right;"|וכן כל הדומה לו | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== <span style=color:green>Scalene Trapezoid</span> ==== | ||
| + | |||
| + | |- | ||
| + | | | ||
| + | :{{#annot:scalene trapezoid|680|JXCu}}132) A trapezoid that has two parallel sides: line AG is 8 cubits long, line BD is 22 cubits long; and two unequal sides: line AB is 15 [cubits] long, and line GD is 13 cubits long. | ||
| + | :It is called scalene trapezoid. | ||
| + | |style="text-align:right;"|‫<ref>115r</ref>קלב <big>קטומה</big> שיש לה ב' קוים נכוחיים קו א"ג ואורכו ח' אמות וקו ב"ז ואורכו כ"ב אמות וב' צלעים שאינם שוות והם קו א"ב ואורכו ט"ו וקו ג"ד ואורכו י"ג אמות<br> | ||
| + | והצורה הזאת נקראת ''{{#annot:trapezoid|596,2207|oONP}}קטומת הראש חלופה{{#annotend:oONP}}''{{#annotend:JXCu}} | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | [[File:כללים במספר - קלב -1.png|thumb|250px|center]] | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | [[File:Kelalim ba-Mispar 132-1.png|thumb|250px|center]] | ||
| + | |- | ||
| + | | | ||
| + | :The area of this shape is found by finding the height, as you did with the first [trapezoid] I described above. | ||
| + | |style="text-align:right;"|וריבוע הצורה הזאת יהיה בהוצאת עמוד ה' כאשר עשית בראשונה שכתבתיה למעלה | ||
| + | |- | ||
| + | | | ||
| + | :In this trapezoid you should find the long segment and the short segment of the base [= the distances between the heights and the meeting points of the longer and shorter sides with the basis] and you do it this way: | ||
| + | |style="text-align:right;"|ואתה צריך בקטומה הזאת לגבל מעמד העמודים ולדעת מעמד הגדול ומעמד הקטון ואתה נוהג בהם המנהג הזה | ||
| + | |- | ||
| + | | | ||
| + | :Square the short side; subtract its square from the square of the long side; take half the difference between them and divide it by the excess of the bottom base over the upper base. If you add the result of division to half the excess of the bottom base, you find the long segment of the height, which is its distance from the longer side. | ||
| + | |style="text-align:right;"|תרבע הצלע הקצורה וגרע מרובעה ממרובע הצלע הארוך והעודף שבהם תקח מחציתו ותחלק אותו על עודף התושבת על הראש ואשר יצא מהחלוקה הזאת אם אתה מוסיף אותו על מחצית עדף התושבת תמצא המעמד הגדול אשר לעמוד והוא מרחקו מן הצלע הארוך | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\left[\frac{1}{2}\sdot\left(\rm{base_{bottom}-base_{top}}\right)\right]+\frac{\frac{1}{2}\sdot\left(\rm{side_1^2-side_2^2}\right)}{\rm{base_{bottom}-base_{top}}}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :If you subtract it from half the excess, you find the short segment of the height, which is its distance from the short side. | ||
| + | |style="text-align:right;"|ואם תגרעינו ממחצית העודף תמצא המעמד הקטן אשר לעמוד והוא מרחקו מן הצלע הקצר | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\left[\frac{1}{2}\sdot\left(\rm{base_{bottom}-base_{top}}\right)\right]-\frac{\frac{1}{2}\sdot\left(\rm{side_1^2-side_2^2}\right)}{\rm{base_{bottom}-base_{top}}}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :In this shape, if you square 13, which is the length of the short side GD, its square is 169. | ||
| + | |style="text-align:right;"|ואם אתה מרבע בצורה הזאת י"ג שהוא אורך צלע ג"ד הקצור יהיה מרובעו ק'ס'ט‫' | ||
| + | |- | ||
| + | | | ||
| + | :Subtract it from the square of AB, which is the long side; 56 remains. | ||
| + | |style="text-align:right;"|פחות אותו ממרובע א"ב שהוא מרובע הצלע הארוך ישאר נ"ו | ||
| + | |- | ||
| + | | | ||
| + | :Take half this number; it is 28. | ||
| + | |style="text-align:right;"|קח מחצית המניין הזה והוא כ"ח | ||
| + | |- | ||
| + | | | ||
| + | :Divide it by 14, which is the excess of the bottom base over the upper base; the quotient is 2. | ||
| + | |style="text-align:right;"|חלק אותו על י"ד שהוא עודף התושבת על הראש תהיה החלוקה ב‫' | ||
| + | |- | ||
| + | | | ||
| + | :If you add 2 to 7, which is half the excess of the bottom base, it is 9 and this is the length of BH, which is the distance of the height from the long side. | ||
| + | |style="text-align:right;"|ואם תוסיף ב' על ז' אשר הוא חצי עדף התושבת יהיה ט' והוא אורך קו ב"ה אשר הוא מרחק העמוד מהצלע הארוך | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle BH &\scriptstyle=\left[\frac{1}{2}\sdot\left(BD-AG\right)\right]+\frac{\frac{1}{2}\sdot\left(AB^2-GD^2\right)}{BD-AG}=\left[\frac{1}{2}\sdot\left(22-8\right)\right]+\frac{\frac{1}{2}\sdot\left(15^2-13^2\right)}{22-8}\\&\scriptstyle=\left(\frac{1}{2}\sdot14\right)+\frac{\frac{1}{2}\sdot\left(15^2-169\right)}{14}=7+\frac{\frac{1}{2}\sdot56}{14}=7+\frac{28}{14}=7+2=9\\\end{align}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :If you subtract 2 from 7, you find the distance of the height from the short side. | ||
| + | :<math>\scriptstyle{\color{blue}{CD=\left[\frac{1}{2}\sdot\left(BD-AG\right)\right]-\frac{\frac{1}{2}\sdot\left(AB^2-GD^2\right)}{BD-AG}=7-2}}</math> | ||
| + | |style="text-align:right;"|ואם תפחות ב' מז' תמצא מרחק העמוד מהצלע הקצר | ||
| + | |- | ||
| + | | | ||
| + | :The proof of this matter is that you examine what I explained above regarding the height of the scalene triangle: | ||
| + | |style="text-align:right;"|והאות על העניין הזה התבונן לך מאשר פירשתי למעלה בעמוד המשולש מתחלף הצלעות | ||
| + | |- | ||
| + | | | ||
| + | :[[File:Kelalim ba-Mispar 132-2.png|thumb|250px|left]] | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :Because, when you subtract the upper base, which is 5, from the bottom base, the remainder is line TC, which is 3 cubits minus a seventh, and this is the excess of the segment of the height of the triangle over the distance of the height of the trapezoid from its short side. | ||
| + | |style="text-align:right;"|כי בהוציאך מן התושבת את הרחב הראש והוא ה' ישאר קו ט"ח ג' אמות פחות שביע והוא עודף מעמד עמוד המשולש על מעמד עמוד הקטומה הקצר | ||
| + | |- | ||
| + | | | ||
| + | :Multiply this excess by the short side of the trapezoid, which is 13; it is 37 and a seventh. | ||
| + | |style="text-align:right;"|והעודף הזה רבע בצלע הקטומה הקצר שהוא י"ג יהיה ל"ז ושביעית | ||
| + | |- | ||
| + | | | ||
| + | :Divide this number by the short segment of the trapezoid, which is 5; the quotient is 7 and 3-sevenths and this is the excess of the side of the triangle over the short side of the trapezoid, which is line GZ that is drawn from the short side. | ||
| + | |style="text-align:right;"|בוא וחלק המספר הזה על מעמד העמוד הקצר בקטומה והוא ה' תהיה החלוקה הזאת ז' וג' שביעיות והוא עודף צלע המשולש על צלע הקטומה הקצרה והוא קו ג"ז היוצא מצד הקצר | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{GZ=\frac{TC\sdot GD}{CD}=\frac{\left(DT-CD\right)\sdot GD}{CD}=\frac{\left(3-\frac{1}{7}\right)\sdot13}{5}=\frac{37+\frac{1}{7}}{5}=7+\frac{3}{7}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :If you want to find the short line AZ, find the long segment of the triangle according to the way you found the short segment. | ||
| + | |style="text-align:right;"|ואם תרצה לדעת קו א"ז הארוך הוצא המעמד הארוך במשולש על העניין שהוצאת הקצר | ||
| + | |- | ||
| + | | | ||
| + | :You find it is 14 and a seventh and it exceeds the long segment by 5 and a seventh. | ||
| + | |style="text-align:right;"|ותמצא אותו י"ד ושביעית והוא מעדיף על מעמד הארוך ה' ושביעית | ||
| + | |- | ||
| + | | | ||
| + | :Multiply it by the long side, which is 15; the product is 77 and a seventh. | ||
| + | |style="text-align:right;"|מנה אותו בצלע הארוך שהוא ט"ו יהיה המניין ע"ז ושביעית | ||
| + | |- | ||
| + | | | ||
| + | :Divide this number by 9, which is the long segment of the trapezoid; the quotient is 8 and 4-sevenths and this is the excess of the side of the triangle over the long side of the trapezoid, which is AZ. | ||
| + | |style="text-align:right;"|חלק המספר הזה על ט' שהוא המעמד הארוך בקטומה תהיה החלוקה ח' וד' שביעיות והוא עודף צלע המשולש על צלע הקטומה הא' הארוך והוא א"ז | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{AZ=\frac{HT\sdot AB}{HB}=\frac{\left(BT-HB\right)\sdot AB}{HB}=\frac{\left[\left(14+\frac{1}{7}\right)-9\right]\sdot 15}{9}=\frac{\left(5+\frac{1}{7}\right)\sdot15}{9}=\frac{77+\frac{1}{7}}{9}=8+\frac{4}{7}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :If you want to find the height of this triangle, multiply the excess of the short segment, which is 3 minus a seventh, by the height of the trapezoid, which is 12. | ||
| + | |style="text-align:right;"|ואם תבוא לדעת עמוד המשולש הזה הוי מונה עודף המעמד הקטן והוא ג' פחות שביע בעמוד הקטומה שהוא י"ב | ||
| + | |- | ||
| + | | | ||
| + | :Then, divide it by the short segment, which is 5. | ||
| + | |style="text-align:right;"|וחלק אותו על מעמד הקטן והוא ה‫' | ||
| + | |- | ||
| + | | | ||
| + | :Or, multiply the excess of the long segment, which is 5 and a seventh, by the height of the trapezoid, which is 12. | ||
| + | |style="text-align:right;"|או הוי מונה עודף המעמד הגדול והוא ה' ושביע בעמוד הקטומה שהוא י"ב | ||
| + | |- | ||
| + | | | ||
| + | :We divide it by the long segment, which is 9. | ||
| + | |style="text-align:right;"|נחלקהו על המעמד הגדול והוא ט‫' | ||
| + | |- | ||
| + | | | ||
| + | :You find both results are the same number, which is 7 minus a seventh, and this is the excess of the height of the triangle over the height of the trapezoid. | ||
| + | |style="text-align:right;"|תמצא שניהם מוציאים אל חשבון אחד והוא ז' פחות שביע והוא עודף עמוד המשולש על עמוד הקטומה | ||
| + | |- | ||
| + | | | ||
| + | :Add it to 12, which is the height of the trapezoid; the total is 19 minus a seventh and this is the length of line ZT, which is the height of the triangle in this shape. | ||
| + | |style="text-align:right;"|הוסף אותו על י"ב שהוא עמוד הקטומה ויהיה הכל י"ט פחות שביע והוא אורך קו ז"ט אשר הוא עמוד המשולש בצורה הזאת | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{ZT=\frac{TC\sdot GC}{CD}+GC=\frac{\left(3-\frac{1}{7}\right)\sdot12}{5}+12=\left(7-\frac{1}{7}\right)+12=19-\frac{1}{7}}}</math> | ||
| + | :<math>\scriptstyle{\color{blue}{ZT=\frac{HT\sdot AH}{BH}+AH=\frac{\left(5+\frac{1}{7}\right)\sdot12}{9}+12=\left(7-\frac{1}{7}\right)+12=19-\frac{1}{7}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :This matter is clear as we said above. | ||
| + | |style="text-align:right;"|‫<ref>116r</ref><big>גליון</big> וזה דבר ברור הוא כאשר אמרנו למעלה | ||
| + | |- | ||
| + | | | ||
| + | :Because, [the ratio of] line HT to line AH is the same as [the ratio of] line BH to the line of the excess of the height of the triangle over the height of the trapezoid, which is AH. | ||
| + | |style="text-align:right;"|כי קו ה"ט בקו א"ה כקו ב"ה בקו עודף עמוד המשולש על עמוד הקטומה שהיה א"ה | ||
| + | |- | ||
| + | | | ||
| + | :Therefore, if we multiply line HT by line AH and divide by line BH, we get the excess. | ||
| + | |style="text-align:right;"|על כן אם נרבע קו ה"ט בקו א"ה ונחלק על קו ב"ה יצא לנו העודף | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== <span style=color:green>Right Trapezoid</span> ==== | ||
| + | |||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :{{#annot:right trapezoid|680,683|Tojz}}133) The third shape is the trapezoid whose upper base is parallel to its lower base and one of its legs is its height. This shape is called "right trapezoid". | ||
| + | :For example: [right] trapezoid ABGD - its upper base AG is 8, its lower base BD is 20, its long leg AB is 15, and its short leg GD is 9 cubits, which is the height of BD. | ||
| + | |style="text-align:right;"|קלג התמונה השלישית הקטומה שראשה נכוחי לתושבתה ואחד מצלעיה עמוד עליה והתמונה הזאת נקראת ''חצי קטומה''<br> | ||
| + | והדמיון לצורה הזאת קטומה שעליה א'ב'ג'ד' וראשה א"ג והיא ח' ותושבתה ב"ד והיא כ' וצלע א"ב הארוך ט"ו וצלע ג"ד הקצור ט' אמות והוא עמוד על תושבת ב"ד{{#annotend:Tojz}} | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | [[File:כללים במספר - קלג.png|thumb|300px|center]] | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | [[File:Kelalim ba-Mispar 133.png|thumb|300px|center]] | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :The calculation of the area of this trapezoid is by adding its upper base to its lower base, then taking its half, and multiplying it by the height; this is the area of the trapezoid. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\left[\frac{1}{2}\sdot\left(\rm{base_{top}+base_{bottom}}\right)\right]\sdot\rm{height}}}</math> | ||
| + | |style="text-align:right;"|וחשבון רבוע הקטומה הזאת הוא באסיפת ראשה אל תושבתה ולקחת מחציתם וימנה בעמוד ויהיה תשבורת הקטומה | ||
| + | |- | ||
| + | | | ||
| + | :In this shape, if you add its upper base to its lower base, their sum is 28 and its half is 14. | ||
| + | |style="text-align:right;"|ואם תאסוף בצורה הזאת ראשה אל תושבתה יהיה כללם כ"ח ומחציתם י"ד | ||
| + | |- | ||
| + | | | ||
| + | :If you multiply this number by the length of the height, which is 9, it is 126 and this is the area of this trapezoid. | ||
| + | :<math>\scriptstyle{\color{blue}{\left[\frac{1}{2}\sdot\left(8+20\right)\right]\sdot9=\left(\frac{1}{2}\sdot28\right)\sdot9=14\sdot9=126}}</math> | ||
| + | |style="text-align:right;"|ואם תרבע המספר הזה באורך העמוד והוא ט' יהיה ק'כ'ו' והוא תשבורת הקטומה הזאת | ||
| + | |- | ||
| + | | | ||
| + | :The proof of this is explained above regarding the complete trapezoid. | ||
| + | |style="text-align:right;"|ואות לזה מפורש למעלה בקטומה הנשלמת | ||
| + | |- | ||
| + | | | ||
| + | :If you want to know its short diagonal, which is AD: | ||
| + | |style="text-align:right;"|ואם תבוא לדעת אלכסונה הקצור והוא א"ד | ||
| + | |- | ||
| + | | | ||
| + | :Square the upper base, which is 8; its square is 64. | ||
| + | |style="text-align:right;"|הוי מרבע את הראש והוא ח' ומרובעו ס"ד | ||
| + | |- | ||
| + | | | ||
| + | :Add to it the square of the height, which is the short side that is 81; together they are 145 and this is the square of the diagonal. | ||
| + | |style="text-align:right;"|ואסוף אליו מרובע העמוד והוא הצלע הקצור והוא פ"א ויהיו שניהם יחד ק'מ'ה' והוא מרובע האלכסון | ||
| + | |- | ||
| + | | | ||
| + | :The root of this number is the length of the short diagonal. | ||
| + | |style="text-align:right;"|וגדר המספר הזה הוא אורך אלכסון הקצור | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{AD=\sqrt{\rm{base_{top}^2+height^2}}=\sqrt{8^2+9^2}=\sqrt{64+81}=\sqrt{145}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :If you want to know the long diagonal: | ||
| + | |style="text-align:right;"|ואם תרצה לדעת אלכסון הארוך | ||
| + | |- | ||
| + | | | ||
| + | :Add the square of the bottom base to the square of the height; they are 481 and this is the square of the long diagonal. | ||
| + | |style="text-align:right;"|אסוף מרובע התושבת למרובע העמוד ויהיו שניהם ת'פ'א' והוא מרובע אלכסון הארוך | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{GB=\sqrt{\rm{base_{bottom}^2+height^2}}=\sqrt{20^2+9^2}=\sqrt{481}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :This calculation is correct, because both angles at point G and point D on side GD are right angles, since this side is perpendicular to the lower base and the upper base that are parallel. | ||
| + | |style="text-align:right;"|ונתכוון לך החשבון הזה מפני ששתי זוויות אשר על נקודת ג' ונקודת ד' מן צלע ג"ד הן ניצבות כי הצלע הזה הוא עמוד על התושבת ועל הראש אשר הם {{#annot:term|825,1821|uvqN}}נכוחיים{{#annotend:uvqN}} | ||
| + | |- | ||
| + | | | ||
| + | :Diagonal AD is the hypotenuse opposite the right angle G. | ||
| + | |style="text-align:right;"|ואלכסון א"ד הוא מיתר לזוית ג' הניצבה | ||
| + | |- | ||
| + | | | ||
| + | :Diagonal GB is the hypotenuse opposite the right angle D. | ||
| + | |style="text-align:right;"|ואלכסון ג"ב הוא מיתר לזוית ד' הניצבה | ||
| + | |- | ||
| + | | | ||
| + | :The square of the hypotenuse opposite the right angle is equal to [the sum of] the squares of the two sides comprising it, as you know. | ||
| + | |style="text-align:right;"|ומרובע מיתר זוית הניצבה הוא שוה לשם מרובעי ב' הצלעות אשר הן מקיפות לה כאשר ידעת | ||
| + | |- | ||
| + | | | ||
| + | :This is your proof of calculating the diagonals in this shape. | ||
| + | |style="text-align:right;"|וזה לך האות על חשבון האלכסונות בצורה הזאת | ||
| + | |- | ||
| + | | | ||
| + | :If you want to complete this trapezoid and draw the top of the triangle from which it is cut off: | ||
| + | |style="text-align:right;"|ואם תרצה להשלים הקטומה הזאת ולהוציא ראש המשולש הנקטמת ממנה | ||
| + | |- | ||
| + | | | ||
| + | :Apply the procedure you learned for the previous trapezoid: | ||
| + | |style="text-align:right;"|עשה כמעשה שלמדת מן הקטומה שלפניה | ||
| + | |- | ||
| + | | | ||
| + | :Find the excess of the lower base over the upper base, which is 12 in this shape. | ||
| + | |style="text-align:right;"|והוא שתהיה יודע עודף התושבת על הראש והוא י"ב בצורה הזאת | ||
| + | |- | ||
| + | | | ||
| + | :If you multiply the upper base by line AB, then divide it by the excess of the lower base, you get the length of the line drawn from the height [of the trapezoid] to the top of the triangle. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\frac{\rm{base_{top}}\times\rm{long\ side_{trapezoid}}}{\rm{base_{bottom}-base_{top}}}}}</math> | ||
| + | |style="text-align:right;"|ואם תמנה הראש בקו א"ב וחלקנו על עודף התושבת יצא לך אורך הקו היוצא מהעמוד אל ראש המשולש | ||
| + | |- | ||
| + | | | ||
| + | :You can apply it the way you learned for the other shapes. | ||
| + | |style="text-align:right;"|ואתה יכול להוציאו למעשה מן הדרך שלמדת בצורות האחרות | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== <span style=color:green>Obtuse Trapezoid</span> ==== | ||
| + | |||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :{{#annot:obtuse trapezoid|680,685,683|dvwi}}134) A trapezoid whose upper base is parallel to its lower base and one of its legs is at obtuse angle to the lower base. | ||
| + | :This trapezoid has two inner heights and one outer height. | ||
| + | :It is an obtuse trapezoid | ||
| + | :For example: [obtuse] trapezoid ABGD - its upper base AG is 14, its lower base BD is 21, its long leg AB is 20, and its short leg GD is 15. | ||
| + | |style="text-align:right;"|קלד <big>קטומה</big> שראשה נכוחי לתושבתה והצלע אחת נופלת על התושבת על זוית נרווחת<br> | ||
| + | ולקטומה הזאת שני עמודים תכונים ועמוד חצוני<br> | ||
| + | והיא ''{{#annot:trapezoid|1105,2209|xzTs}}קטומה מתמוטטת{{#annotend:xzTs}}''<br> | ||
| + | והדמיון קטומה שעליה א'ב'ג'ד' וקו א"ג ראשה י"ד וקו ב"ד התושבת כ"א וצלע א"ב הארוך כ' וצלע ג"ד הקצור ט"ו{{#annotend:dvwi}} | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | [[File:כללים במספר - קלד -1.png|thumb|350px|center]] | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | [[File:Kelalim ba-Mispar 134-1.png|thumb|325px|center]] | ||
| + | |- | ||
| + | | | ||
| + | :You know the area of this trapezoid by extracting its heights: | ||
| + | |style="text-align:right;"|ואתה יודע תשבורת הקטומה הזאת בהוצאת עמודיה | ||
| + | |- | ||
| + | | | ||
| + | :First, you should find [the distance between the height and the meeting points of the sides with the basis]: | ||
| + | |style="text-align:right;"|ואתה צריך תחלה להגביל מעמד העמוד | ||
| + | |- | ||
| + | | | ||
| + | :It is by that you subtract the upper base, which is 14, from the bottom base, which is 21; you are left with 7 and this is the excess of the bottom base. | ||
| + | |style="text-align:right;"|והוא שתהיה פוחת הראש והוא י"ד מן התושבת והוא כ"א ישאר בידך ז' והוא עודף התושבת | ||
| + | |- | ||
| + | | | ||
| + | :Square this excess and add its square to the square of the short leg; you find [the sum of] these two squares is 274. | ||
| + | |style="text-align:right;"|ובוא ורבע העודף הזה ואסוף מרובעו אל מרובע הצלע הקצור ותמצא ב' מרובעים אילו ר'ע'ד‫' | ||
| + | |- | ||
| + | | | ||
| + | :Subtract this number from the square of the short leg, which is 400; you are left with 126. | ||
| + | |style="text-align:right;"|הוצא המספר ממרובע הצלע הארוך שהוא ת' ישאר בידך ק'כ'ו‫' | ||
| + | |- | ||
| + | | | ||
| + | :Take its half, which is 63, and divide it by 7, which is the excess of the bottom base. | ||
| + | |style="text-align:right;"|קח המחצית ‫<ref>116v</ref>והוא ס"ג חלק אותו על ז' והוא עודף התושבת | ||
| + | |- | ||
| + | | | ||
| + | :The total is 16, which is the [distance between the height and the meeting point of the longer side AB with the basis]. | ||
| + | :<math>\scriptstyle{\color{blue}{HB=16}}</math> | ||
| + | |style="text-align:right;"|ויהיה הכל י"ו והוא גבול מעמד העמוד מנגד קו א"ב הארוך | ||
| + | |- | ||
| + | | | ||
| + | :The 9 you have found is short segment. | ||
| + | |style="text-align:right;"|ואלו הט' אשר מצאת הם המרחק הקצר | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle CD &\scriptstyle=\frac{\frac{1}{2}\sdot\left[AB^2-\left[\left(BD-AG\right)^2+GD^2\right]\right]}{BD-AG}=\frac{\frac{1}{2}\sdot\left[20^2-\left[\left(21-14\right)^2+15^2\right]\right]}{21-14}=\frac{\frac{1}{2}\sdot\left[20^2-\left(7^2+15^2\right)\right]}{7}\\&\scriptstyle=\frac{\frac{1}{2}\sdot\left(400-274\right)}{7}=\frac{\frac{1}{2}\sdot126}{7}=\frac{63}{7}=9\\\end{align}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :So is the distance of the outer height GC from line BD, which is the lower base, and from the middle height DT, which falls on point D. | ||
| + | |style="text-align:right;"|וכן מרחק העמוד שעליו ג"ח אשר הוא יוצא חוצה מן קו ב"ד התושבת וכן מרחק העמוד התיכוני הנופל על נקודת ד' שעליו ד"ט | ||
| + | |- | ||
| + | | | ||
| + | :If you want to know the length of the height: | ||
| + | |style="text-align:right;"|ואם תבוא לדעת אורך העמוד | ||
| + | |- | ||
| + | | | ||
| + | :Square the segment you want. | ||
| + | |style="text-align:right;"|הוי מרבע את מעמד שתרצה | ||
| + | |- | ||
| + | | | ||
| + | :If you square the long segment, which is 16, subtract it from the square of the long side, which is 20. | ||
| + | |style="text-align:right;"|ואם [אתה] מרבע המעמד הארוך שהוא י"ו הוציאנו ממרובע הצלע הארוך אשר הוא כ‫' | ||
| + | |- | ||
| + | | | ||
| + | :If you square the short segment, subtract it from the square of the short side. | ||
| + | |style="text-align:right;"|ואם תרבע המעמד הקצור הוציאנו ממרובע הצלע הקצר | ||
| + | |- | ||
| + | | | ||
| + | :The remainder in both calculations is 144 and this is the square of the height. | ||
| + | |style="text-align:right;"|ויהיה המותר מן כל אחד משני החשבונים האלה ק'מ'ד' והוא מרובע העמוד בק | ||
| + | |- | ||
| + | | | ||
| + | :Its root is 12 and this is the length of the height. | ||
| + | |style="text-align:right;"|וגדרו י"ב והוא אורך העמוד | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{AH=\sqrt{AB^2-HB^2}=\sqrt{20^2-16^2}=\sqrt{144}=12}}</math> | ||
| + | :<math>\scriptstyle{\color{blue}{GC=\sqrt{GD^2-CD^2}=\sqrt{15^2-9^2}=\sqrt{144}=12}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :The area of this trapezoid is [found] by multiplying the height by [the sum of] half the upper base and half the lower base, as you did for the previous trapezoids. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\left[\frac{1}{2}\sdot\left(\rm{base_{top}+base_{bottom}}\right)\right]\sdot\rm{height}}}</math> | ||
| + | |style="text-align:right;"|ותשבורת הקטומה הוא ברבוע העמוד בחצי הראש ובחצי התושבת כאשר עשית בשאר הקטומות שלפניה | ||
| + | |- | ||
| + | | | ||
| + | :The area is 210. | ||
| + | :<math>\scriptstyle{\color{blue}{\left[\frac{1}{2}\sdot\left(14+21\right)\right]\sdot12=210}}</math> | ||
| + | |style="text-align:right;"|והתשבורת הוא ר"י | ||
| + | |- | ||
| + | | | ||
| + | :The proof for this calculation in which you extract the height is as the proof that I taught you for the scalene trapezoid. | ||
| + | |style="text-align:right;"|והאות על החשבון הזה שהוצאת בו את העמוד הוא מעניין האות שהורתיך בקטומה שאין צלעותיה שוות | ||
| + | |- | ||
| + | | | ||
| + | :Because, when you subtract the upper base from the lower base, and you are left with 7, this line is a side of an obtuse triangle, whose one side is 7 and the other is 15, and they are the sides comprising the obtuse angle. | ||
| + | |style="text-align:right;"|כי בהוציאך הראש מהתושבת ונשארו בידך ז' היה הקו ההוא צלע ל{{#annot:triangle|1105,2184|iUfN}}משולש מרויח הזויות{{#annotend:iUfN}} א' שצלעו האחת ז' והשינית ט"ו והם הצלעות המקיפות זוית הנרווחת | ||
| + | |- | ||
| + | | | ||
| + | :The hypotenuse of this angle is 20 cubits, which is the length of line AB in this trapezoid. | ||
| + | |style="text-align:right;"|ומיתר הזוית הזאת הוא כ' אמה אשר הוא אורך קו א"ב מן הקטומה הזאת | ||
| + | |- | ||
| + | | | ||
| + | :If you draw an outer height in this obtuse triangle, you find its position outside, as you find in this shape. | ||
| + | |style="text-align:right;"|ואם אתה מוציא במשולש הזה המרויח הזויות עמוד שיהיה נופל חוצה אתה מוצא מעמדו חוצה כאשר מצאת בחשבונך בצורה הזאת | ||
| + | |- | ||
| + | | | ||
| + | :[[File:Kelalim ba-Mispar 134-2.png|thumb|200px|left]] | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :You can find the long diagonal of the trapezoid, if you add the segment of the outer height to the lower base, then square the total. | ||
| + | |style="text-align:right;"|ואתה יכול להוציא {{#annot:term|1106,1878|HaZi}}אלכסון{{#annotend:HaZi}} הקטומה הארוך אם אתה מאסף אל התושבת מעמד העמוד החיצוני וכולל את הכל ומרבע אותו | ||
| + | |- | ||
| + | | | ||
| + | :As if you add 21, which is the length of the lower base in this shape, to 9, which is the length of the segment of the outer height; the total is 30. | ||
| + | |style="text-align:right;"|כאילו היית מאסף בצורה הזאת כ"א שהוא אורך התושבת אל ט' שהוא אורך מעמד העמוד חוצה ויהיה הכל ל‫' | ||
| + | |- | ||
| + | | | ||
| + | :You square this number and add the square of the height to it; the total is 1044 and this is the square of the long diagonal. | ||
| + | |style="text-align:right;"|ואתה מרבע המניין הזה ומוסיף עליו מרובע העמוד ויהיה הכל אלף ומ"ד והוא מרובע האלכסון הארוך | ||
| + | |- | ||
| + | | | ||
| + | :The root of this number is the length of the diagonal from point G to point B in this trapezoid. | ||
| + | |style="text-align:right;"|וגדר המספר הזה הוא אורך האלכסון בקטומה הזאת מנקודת ג' אל נקודת ב‫' | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{GB=\sqrt{\left(BD+CD\right)^2+GC^2}=\sqrt{\left(21+9\right)^2+12^2}=\sqrt{30^2+12^2}=\sqrt{1044}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :If you want to find the short diagonal going from A to D: | ||
| + | |style="text-align:right;"|ואם תרצה להוציא אלכסון הקצור היוצא מן א' ועד ד‫' | ||
| + | |- | ||
| + | | | ||
| + | :Add the square of the height to the square of the excess of the lower base over the long segment, which is 5 in this shape; [the sum of] the two squares is 169 and this is the square of the short diagonal. | ||
| + | |style="text-align:right;"|תאסוף אל מרובע העמוד מרובע עודף התושבת על המעמד הארוך והוא בצורה הזאת ה' ויהיו שני המרובעים ק'ס'ט' והוא מרובע האלכסון הקצור | ||
| + | |- | ||
| + | | | ||
| + | :Its root is the length of the diagonal. | ||
| + | |style="text-align:right;"|וגדרו הוא אורך האלכסון | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{AD=\sqrt{\left(BD-HB\right)^2+AH^2}=\sqrt{\left(21-16\right)^2+12^2}=\sqrt{5^2+12^2}=\sqrt{169}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :You can understand the proof of this matter from the proofs above, when you look at right triangles formed by these diagonals, the sides of the trapezoid, and the heights, as I showed you above. | ||
| + | |style="text-align:right;"|והאות אל העניין הזה אתה יכול להבין מהאותות אשר למעלה אם אתה מביט אל ה{{#annot:triangle|1104,1341|1xD2}}משולשים מוצבי זוית{{#annotend:1xD2}} אשר הם מתיילדים מהאלכסונות האלה עם צלעי הקטומה והעמודים כאשר הראיתך למעלה | ||
| + | |- | ||
| + | | | ||
| + | :The short diagonal of this shape can be also the height of the trapezoid itself. | ||
| + | |style="text-align:right;"|ויכול הוא בצורה הזאת שיהיה אלכסון הקצור הוא עמוד בקטומה בעצמו | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | [[File:כללים במספר - קלד -3.png|thumb|250px|center]] | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | [[File:Kelalim ba-Mispar 134-3.png|thumb|200px|center]] | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:obtuse trapezoid|683|RYHe}}As if the length of the upper base of the trapezoid [AG] is 9 cubits, its lower base [BD] is 16, and the size of the other two sides is the same as in the first shape. | ||
| + | |style="text-align:right;"|כאילו היה בצורה הזאת אורך ראש הקטומה ט' אמות ותושבתה י"ו ומניין ב' הצלעות הנשארות המניין אשר היה להם בצורה הראשונה{{#annotend:RYHe}} | ||
| + | |- | ||
| + | | | ||
| + | :You find the height in this second shape falling from point A to point D and this height is the length of the short diagonal [= AD]. | ||
| + | |style="text-align:right;"|והיית מוצא בצורה הזאת השינית העמוד נופל מנקודת א' אל נקודת ד' ויהיה העמוד ההוא אורך האלכסון הקצר | ||
| + | |- | ||
| + | | | ||
| + | :The long diagonal going from G to B is the root of 769, which is [the sum of] the square of the height AD with the square of line AG and line BD that are the upper base and the lower base of the trapezoid together in one line. | ||
| + | |style="text-align:right;"|והאלכסון הארוך אשר הוא יוצא מג' עד ב' הוא גדר ת'ש'ס'ט' אשר הוא מרבע עמוד א"ד ‫<ref>117r</ref>עם מרובע קו א"ג עם קו ב"ד אשר ראש הקטומה ותושבת שניהם יחד בקו אחד | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{GB=\sqrt{\left(AG+BD\right)^2+AD^2}=\sqrt{\left(16+9\right)^2+12^2}=\sqrt{769}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :You understand the proofs of all this calculation in place if you examine it properly. | ||
| + | |style="text-align:right;"|ואתה מבין אותות כל החשבון הזה על מכונו אם אתה מעיין בה עיון יפה | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== <span style=color:green>Quadrangle which has no parallel sides</span> ==== | ||
| + | |||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :{{#annot:quadrangle|680|pQjb}}135) The second type of the rest of the shapes that have four sides are all the quadrangles that none of their sides is parallel to another side. | ||
| + | |style="text-align:right;"|קלה <big>המין</big> השני הנשאר מן התמונות אשר יש להם ד' צלעות הוא כל ''מרובע שאין אחת מצלעותיו נכוחי לצלע אחרת''{{#annotend:pQjb}} | ||
| + | |- | ||
| + | | | ||
| + | :You find the areas of these shapes only by finding the areas of the triangles into which they are divided. | ||
| + | |style="text-align:right;"|ובתמונות האלו אי אתה מוצא רבועם אלא מרבוע המשולשי' אשר הם נחלקי' עליהם | ||
| + | |- | ||
| + | | | ||
| + | :You can divide every quadrangle in the world into two triangles. | ||
| + | |style="text-align:right;"|וכל מרובע בעולם אתה יכול לחלקו לשני משולשין | ||
| + | |- | ||
| + | | | ||
| + | :It is known that whoever divides it into these two triangles, then sums up their areas, finds the area of the quadrangle they are parts of. | ||
| + | |style="text-align:right;"|וידוע הוא כי המשבר שני המשולשי' ההם ומצרף תשברתם ימצא התשבורת המרובע אשר הם חלקיו | ||
| + | |- | ||
| + | | | ||
| + | :This way you can find the area of all quadrangles of different types, whose sides are equal or parallel, from the areas of the triangles into which they are divided. | ||
| + | |style="text-align:right;"|ועל הענין הזה תוכל למצא התשבורת כל מרובע על חלופי מיניהן מן השוים בצלעותיו והנכוחיים מתשבורת המשולשים שהן נחלקים עליהם | ||
| + | |- | ||
| + | | | ||
| + | :But, you can find the area of the quadrangles whose sides are equal or parallel in another method and you do not need to find the areas of the triangles into which they are divided. | ||
| + | |style="text-align:right;"|אלא שהמרובעים השוים בצלעותיו והנכוחיים בהם אתה יכול להגיע אל תשברתן מדרך אחרת ואינך צריך לרבע בהן את המשולשים הנחלקים עליהם | ||
| + | |- | ||
| + | | | ||
| + | :You can find the area of the other quadrangles that do not have parallel sides only by [calculating] the areas of the triangles into which they are divided. | ||
| + | |style="text-align:right;"|ושאר המרובעים הנפתלים ואין בהם צלע {{#annot:term|825,1821|Jegs}}נכוחי{{#annotend:Jegs}} ובצלע אחד אי אתה יכול למצוא תשברתם אלא מתשבורת המשולש אשר יחלקו עליהם | ||
| + | |- | ||
| + | | | ||
| + | *I give you an example for this case and it will be enough for you for the other shapes. This example is of the shape whose explanation I have completed. | ||
| + | |style="text-align:right;"|ואני נותן לך דמיון בעניין זה ויהיה מספיק לך בשאר הצורות והדמיון הזה הוא מהצורה שהשלמתי פירושה | ||
| + | |- | ||
| + | | | ||
| + | | | ||
| + | :[[File:Kelalim ba-Mispar 135-1.png|thumb|200px|left]] | ||
| + | |- | ||
| + | | | ||
| + | :This is the fourth obtuse trapezoid [ABGD] in which side AG is 14 cubits, side BD is 21 cubits, side AB is 20 cubits, and side GD is 15 cubits. | ||
| + | |style="text-align:right;"|והיא הקטומה הרביעית ה{{#annot:trapezoid|1105,2209|Bzov}}מתמוטטת{{#annotend:Bzov}} אשר צלע א"ג היה בה י"ד אמה וצלע ב"ד כ"א אמה וצלע א"ב כ' אמות וצלע ג"ד ט"ו אמה | ||
| + | |- | ||
| + | | | ||
| + | :You find that the short diagonal in this trapezoid is 13 cubits, as we calculated above. | ||
| + | :<math>\scriptstyle{\color{blue}{AD=13}}</math> | ||
| + | |style="text-align:right;"|ומצאת האלכסון הקצור בקטומה הזאת כאשר חשבונו למעלה י"ג אמה | ||
| + | |- | ||
| + | | | ||
| + | :When you measure it, you find the area [of the quadrangle] is greater or smaller [than the area of the given trapezoid]. | ||
| + | |style="text-align:right;"|וכשמדדת אותו אתה מרבע לא מצאת בו המידה הזאת אלא יותר ממנה או פחות ממנה | ||
| + | |- | ||
| + | | | ||
| + | :We say that if you find that diagonal BG is 16 cubits, you know that this shape is not a trapezoid, because none of its sides is parallel to the other, for if they were parallel, its long or short diagonals would have to be exactly the same as they are in the [given] trapezoid. | ||
| + | |style="text-align:right;"|ואנו מוסרין כאילו מצאת אותה יתר ב"ג י"ו אמה ונודע לך מזה כי אין הצורה הזאת קטומת הראש כי אין אחת מצלעיה {{#annot:term|825,1821|E7Zk}}נכוחית{{#annotend:E7Zk}} עם צלע אחרת לפי אם היו נרוחיות לא היה אלכסונה הארוך או הקצר מתחלף מחשבונו שהיא בקטומה | ||
| + | |- | ||
| + | | | ||
| + | :[[File:Kelalim ba-Mispar 135-2.png|thumb|200px|left]] | ||
| + | |[[File:כללים במספר - קלה -2.png|thumb|200px]] | ||
| + | |- | ||
| + | | | ||
| + | :Therefore, you should find the areas of the two triangles into which the diagonal you draw divides this quadrangle; they are triangles ADB and ADG, the sides of each are known. | ||
| + | :<math>\scriptstyle{\color{blue}{ABGD=\triangle_{ADB}+\triangle_{ADG}}}</math> | ||
| + | |style="text-align:right;"|ומפני זה אתה צריך לרבע שני המשולשים אשר נחלק עליהן המרבע הזה באלכסון שהוצאת בו והוא שני משולשי א'ד'ב' וא'ד'ג' וכל אחד מהם צלעותיו ידועות | ||
| + | |- | ||
| + | | | ||
| + | :You can find their areas by finding their heights, as you learned regarding the area of triangles. | ||
| + | |style="text-align:right;"|ואתה יכול להגיע אל ריבועם בהוצאת העמודים כאשר למדת בריבוע המשולשים | ||
| + | |- | ||
| + | | | ||
| + | :If you apply the procedure on them, you find the area of triangle ADB is 96 and a third minus something. | ||
| + | :<math>\scriptstyle{\color{blue}{\triangle_{ADB}=96+\frac{1}{3}-\ldots}}</math> | ||
| + | |style="text-align:right;"|ואם אתה נוהג בהם המנהג הזה תמצא תשבורת משולש א'ד'ב' מהן צ"ו ושליש פחות משהו | ||
| + | |- | ||
| + | | | ||
| + | :The area of triangle ADG is 150 and 2-thirds minus something. | ||
| + | :<math>\scriptstyle{\color{blue}{\triangle_{ADG}=150+\frac{2}{3}-\ldots}}</math> | ||
| + | |style="text-align:right;"|ומשולש א'ד'ג' תהיה תשברתו ק"נ וב' שלישיים פחות משהו | ||
| + | |- | ||
| + | | | ||
| + | :The whole area in this case is 247 minus something. | ||
| + | :<math>\scriptstyle{\color{blue}{ABGD=247-\ldots}}</math> | ||
| + | |style="text-align:right;"|ויהיה כל התשבורת בעניין הזה ר'מ'ז' פחות משהו | ||
| + | |- | ||
| + | | | ||
| + | :We have found the area of the obtuse trapezoid, but we find that the area of this shape is greater, because its diagonal is greater. | ||
| + | |style="text-align:right;"|ואנו מצאנו רבועו בקטומה המתמוטטת ה' ונמצא הריבוע עודף בתמונה הזאת מפני עדיפת האלכסון | ||
| + | |- | ||
| + | | | ||
| + | :If the diagonal were smaller, the area would have been smaller. | ||
| + | |style="text-align:right;"|ואילו היה האלכסון מתמעט היה הריבוע פוחת | ||
| + | |- | ||
| + | | | ||
| + | :From this case you can deduce a general rule for quadrangles: | ||
| + | |style="text-align:right;"|ומהעניין הזה אתה יכול למסור לך גדול במרובעת | ||
| + | |- | ||
| + | | | ||
| + | :I say that the quadrangle whose diagonal you are drawing is divided into two triangles. | ||
| + | |style="text-align:right;"|ואומר מרובע אשר אתה מוציא את אלכסונו נחלק לב' משולשים | ||
| + | |- | ||
| + | | | ||
| + | :If you draw the height in both triangles, then sum the two heights, take half [their sum] and multiply that half by the whole diagonal, you find the area of the quadrangle. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\left[\frac{1}{2}\sdot\left(\rm{height_1+height_2}\right)\right]\sdot\rm{diagonal}}}</math> | ||
| + | |style="text-align:right;"|אם אתה מוציא עמוד של האלכסון בשני המשולשין ותאסוף את שני העמודים ותקח את מחציתם ותצרף המחצית הזה במניין כל האלכסון אתה מוציא תשבורת המרבע | ||
| + | |- | ||
| + | | | ||
| + | :Or, multiply [the sum of] the two heights by half the diagonal and you will also find the area of the quadrangle. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\left(\rm{height_1+height_2}\right)\sdot\left(\frac{1}{2}\sdot\rm{diagonal}\right)}}</math> | ||
| + | |style="text-align:right;"|או תצרף שני העמודים במחצית האלכסון וגם בא תמצא תשבורת המרובע | ||
| + | |- | ||
| + | | | ||
| + | :I do not need to give you an example for this calculation, because you can understand it from the examples of finding the area of triangles and I do not need to elaborate the explanation of the quadrangle further than that. | ||
| + | |style="text-align:right;"|ואיני צריך להביא לך ‫<ref>117v</ref>להביא לך משל מן החשבון הזה מפני שאתה מבין אותו מהדמיונות אשר במשיחת המשולשין ואיני נזקק להאריך בפירוש המרובעי' יותר מזה | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | == <span style=color:green>Additional Problems</span> == | ||
| + | |||
| + | | | ||
| + | |- | ||
| + | !<span style=color:green>Motion Problem - Pursuit</span> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :{{#annot:two men|657|ZNBI}}136) If it is said: A man is walking ten miles a day. | ||
| + | :Another [man] is walking one mile on the first day, two miles on the second day, three miles on the third day, and so on he goes on walking in each day [one mile more]. | ||
| + | :In how many days will [the total distance each of them walked] be equal? | ||
| + | :<math>\scriptstyle10X=\sum_{i=1}^x i</math> | ||
| + | |style="text-align:right;"|קלו <big>אם</big> יאמר הרי אדם שהוא מהלך י' מלים בכל יום<br> | ||
| + | ואחר הוא מהלך ביום ראשון א' מיל וביום שני ב' מילים וביום השלישי ג' ומוסיף והולך בכל יום<br> | ||
| + | בכמה ימים יהיו בשוה{{#annotend:ZNBI}} | ||
| + | |- | ||
| + | | | ||
| + | :<span style=color:red>[See problem 64 above]</span> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :Double the miles of the one who walks the same distance [each day]; they are 20. | ||
| + | |style="width:45%;text-align:right;"|תכפול המילים שמהלך הקבוע יהיו כ‫' | ||
| + | |- | ||
| + | | | ||
| + | :Subtract one from them; 19 remains. So, in 19 days they will [walk] the same [distance]. | ||
| + | :<math>\scriptstyle{\color{blue}{x=\left(2\sdot10\right)-1=20-1=19}}</math> | ||
| + | |style="text-align:right;"|וגרע מהם אחד ישארו י"ט הרי שבי"ט ימים יהיו בשוה | ||
| + | |- | ||
| + | | | ||
| + | :Always double [the miles] of the one who walks a fixed distance [each day] and subtract one from them. | ||
| + | |style="text-align:right;"|וכן לעולם תכפול שמהלך הקבוע ותגרע מהם אחד | ||
| + | |- | ||
| + | | | ||
| + | :{{#annot:two men|657|GFZ9}}137) if it is said: A man is walking ten miles a day. | ||
| + | :Another [man] is walking one mile on the first day, three miles on the second day, five miles on the third day, and so on he goes on walking in each day an odd number of miles. | ||
| + | :In how many days will [the total distance each of them walked] be equal? | ||
| + | :<math>\scriptstyle10X=\sum_{i=1}^x \left(2i-1\right)</math> | ||
| + | |style="text-align:right;"|קלז <big>ואם</big> יאמר הרי אדם שהוא מהלך י' מילים בכל יום<br> | ||
| + | והאחר מהלך ביום אחד א' מיל וביום שני ג' וביום שלישי ה' וביום רבעי ז' ומוסיף והולך בכל יום חשבון נפרד<br> | ||
| + | בכמה יעמדו בשוה{{#annotend:GFZ9}} | ||
| + | |- | ||
| + | | | ||
| + | :Know that as the number of miles of the one who walks a fixed distance each day so is the number of days in which they will [walk] the same [distance]. | ||
| + | |style="text-align:right;"|דע כי כמספר המילים שמהלך הקבוע ליום כן מספר הימים שיעמדו בשוה | ||
| + | |- | ||
| + | | | ||
| + | :If the one [who walks the same distance each day] walks 10 miles each day, they will [walk] the same [distance] in 10 days. | ||
| + | :<math>\scriptstyle{\color{blue}{x=10}}</math> | ||
| + | |style="text-align:right;"|ואם הקבוע מהלך י' מילים ביום בי' ימים יעמדו בשוה | ||
| + | |- | ||
| + | | | ||
| + | :If he walks 9 miles a day, they will [walk] the same [distance] in 9 days. | ||
| + | :<math>\scriptstyle{\color{blue}{x=9}}</math> | ||
| + | |style="text-align:right;"|ואם מהלך ט' מילים ביום בט' ימים יעמדו בשוה וכה"ל | ||
| + | |- | ||
| + | | | ||
| + | :{{#annot:two men|657|FF3W}}138) A man is walking ten miles a day. | ||
| + | :Another [man] is walking two miles on the first day, four miles on the second day, six miles on the third day, and so on he goes on walking in each day an even number of miles. | ||
| + | :In how many days will [the total distance each of them walked] be equal? | ||
| + | :<math>\scriptstyle10X=\sum_{i=1}^x 2i</math> | ||
| + | |style="text-align:right;"|קלח <big>וכן</big> אדם שמהלך בכל יום י' מילים<br> | ||
| + | והאחר מהלך ביום ראשון ב' וביום שני ד' וביום שלשי ו' ומוסיף והולך בכל יום מספר זוג כגון בדוח"י י"ב י"ד י"ו<br> | ||
| + | בכמה ימים יעמדו בשוה{{#annotend:FF3W}} | ||
| + | |- | ||
| + | | | ||
| + | :Subtract one from the miles of the one who walks a fixed distance [each day], which are 10; they are 9. So, in 9 days they will [walk] the same [distance]. | ||
| + | :<math>\scriptstyle{\color{blue}{x=10-1=9}}</math> | ||
| + | |style="text-align:right;"|תחסר אחד מן המילים שמהלך הקבוע שהם י' יהיו ט' הרי שבט' ימים יעמדו בשוה | ||
| + | |- | ||
| + | | | ||
| + | :Always subtract one from the number of miles. The same for everything similar to it. | ||
| + | |style="text-align:right;"|וכן לעולם תחסר אחד ממספר המילים וכן כל הדומה לו | ||
| + | |- | ||
| + | !<span style=color:green>Triangulation Problem - Tree</span> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :{{#annot:broken tree|655|4r4Q}}Question: a tree is ten cubits tall. | ||
| + | :One day a strong wind blew and broke it into two parts, so that the top of the tree snapped and bent from the breaking spot to the ground. | ||
| + | :The distance between the top of the tree and its roots was five cubits. | ||
| + | :How many cubits are there from the top of the tree to the breaking spot? | ||
| + | :<math>\scriptstyle X^2=\left(10-X\right)^2+5^2</math> | ||
| + | |style="text-align:right;"|<big>שאלה</big> אילן שגובהו י' אמות<br> | ||
| + | ובא רוח חזק ושברתו לשנים חלקים ולא נערכו חלקיו אך נטז ממקו' השבירה והגיע ראשו לארץ<br> | ||
| + | והרוחק שבין ראשו לשרשו ה' אמות<br> | ||
| + | כמה אמות מראש האילן עד מקום השבירה{{#annotend:4r4Q}} | ||
| + | |- | ||
| + | | | ||
| + | :The answer: divide the square of the distance from the roots of the tree to its top, which is 25 in our example, by the height of the tree, which is 10; the result is 2 and a half. | ||
| + | |style="text-align:right;"|תשובה תחלק מרובע הרוחק שמשרש האילן לראש שהם כ"ה במשלנו על גובה האילן שהם י' ויצאו ב' וחצי | ||
| + | |- | ||
| + | | | ||
| + | :We add it to the 10, then take a half [of the sum], which is 6 and a quarter and this is the measure from the top of the tree to the breaking spot. | ||
| + | |style="text-align:right;"|נחברם עם הי' ונקח חציים שהם ו' ורביע וזהו כמות האילן עד מקום השבירה | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | :<math>\scriptstyle{\color{blue}{x=\frac{1}{2}\sdot\left(10+\frac{5^2}{10}\right)=\frac{1}{2}\sdot\left(10+\frac{25}{10}\right)=\frac{1}{2}\sdot\left[10+\left(2+\frac{1}{2}\right)\right]=6+\frac{1}{4}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :The complement to ten, which is 3 and 3-quarters, is the number of cubits from the breaking spot to the foot of the tree. | ||
| + | :<math>\scriptstyle{\color{blue}{10-x=10-\left(6+\frac{1}{4}\right)=3+\frac{3}{4}}}</math> | ||
| + | |style="text-align:right;"|והנשאר עד תשלום הי' שהם ג' וג' רביעיות הם כמות האמות שממקום השבירה עד שרש האילן | ||
| + | |- | ||
| + | | | ||
| + | === <span style=color:green>Additional Problems - MS Mantova</span> === | ||
| + | | | ||
| + | |- | ||
| + | !<span style=color:green>Multiple Quantities - Boys Sharing Property of Their Father</span> | ||
| + | | | ||
|- | |- | ||
| | | | ||
| − | + | *{{#annot:sons|652|yRsW}}A man who had sons, their number is unknown, said to one of them: go to the box and take one peraḥ for yourself, and a tithe of the remaining. | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | *{{#annot:sons|652|yRsW}}A man who had sons, their number is unknown, said to one of them: take one peraḥ for yourself, and a tithe of the remaining. | ||
:To the second he said: take two peraḥim and a tithe of the remaining. | :To the second he said: take two peraḥim and a tithe of the remaining. | ||
:And so [he said] to the third [son] and the fourth [son] and the fifth [son] until the last [son]. | :And so [he said] to the third [son] and the fourth [son] and the fifth [son] until the last [son]. | ||
| Line 5,566: | Line 7,392: | ||
:They went and took [the peraḥim] and found out that they all took the same share equally, the number of the peraḥim corresponded to the number of the boys. | :They went and took [the peraḥim] and found out that they all took the same share equally, the number of the peraḥim corresponded to the number of the boys. | ||
:How many were the sons and how many were the peraḥim? | :How many were the sons and how many were the peraḥim? | ||
| − | + | |style="text-align:right;"|‫<ref>Mantova 79r</ref>אדם שהיו לו בנים ולא נודע מספרם<br> | |
| − | |style="text-align:right;"|אדם שהיו לו בנים ולא נודע מספרם<br> | + | ואמר לאחד לך בארגז וקח לך פרח אחד והעשירית מכל הנשאר<br> |
| − | ואמר לאחד לך | ||
ולשני אמ' קח ב' פרחים והעשירית מכל הנשאר<br> | ולשני אמ' קח ב' פרחים והעשירית מכל הנשאר<br> | ||
וכן לשלישי וכן לרביעי ולחמשי עד האחרון<br> | וכן לשלישי וכן לרביעי ולחמשי עד האחרון<br> | ||
| Line 5,574: | Line 7,399: | ||
הלכו ולקחו ומצאו שכולם לקחו בשוה וכמספר הבנים כך מספר הפרחים שיבאו לכל אחד<br> | הלכו ולקחו ומצאו שכולם לקחו בשוה וכמספר הבנים כך מספר הפרחים שיבאו לכל אחד<br> | ||
כמה היו הבנים וכמה היו הפרחי‫'{{#annotend:yRsW}} | כמה היו הבנים וכמה היו הפרחי‫'{{#annotend:yRsW}} | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | :<math>\scriptstyle1+\left[\frac{1}{10}\sdot\left(X-1\right)\right]=2+\frac{1}{10}\sdot\left[X-\left[\left[1+\left[\frac{1}{10}\sdot\left(X-1\right)\right]\right]+2\right]\right]</math> | ||
| + | |- | ||
| + | | | ||
| + | :According to the calculation the fraction is a tenth. | ||
| + | |style="text-align:right;"|לפי חשבון השבור [.....] הוא עשירית | ||
| + | |- | ||
| + | | | ||
| + | :Subtract 1 from ten; 9 remains, so the sons are 9. | ||
| + | :<math>\scriptstyle{\color{blue}{10-\left(\frac{1}{10}\sdot10\right)=10-1=9}}</math> | ||
| + | |style="text-align:right;"|תפחות א' מעשרה ישארו ט' הרי שט' היו הבנים | ||
|- | |- | ||
| | | | ||
| − | ::the | + | :Then, say: 9 times 9 is 81 and so are the peraḥim. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{9\sdot9=81}}</math> |
| − | + | |style="text-align:right;"|אמור אחר זה ט' פעמי' ט' הם פ"א וככה היו הפרחים | |
|- | |- | ||
| | | | ||
| − | : | + | :If they take as their father commanded, each has an equal share. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואם יקחו כאשר צוה אביהם יהיה לכל אחד חלק שוה |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Likewise for any number you wish, do the same: subtract 1 from the denominator of the fraction; this is the number of the sons. Square it; this is the number of the peraḥim. As the number of the sons so is the number of peraḥim the each gets. |
| + | |style="text-align:right;"|וכן לכל חשבון שתרצה עשה כן שתפחות א' מהחשבון השבור והוא חשבון הבנים ותרבע אותו והוא חשבון הפרחי' וכמספר הבנים יהיו ‫<ref>Mantova 79v</ref>מספר הפרחים שיבא לכל אחד ודו"ק | ||
|- | |- | ||
| − | + | !<span style=color:green>MS Mantova; MS Amsterdam: Multiple Quantities - Boys Selling Cubits of a Cloth</span> | |
| | | | ||
|- | |- | ||
| Line 5,602: | Line 7,440: | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''False Position:'''</span> First, I say that a quarter, a fifth and a sixth are found in 60. | |
|style="text-align:right;"|אומר תחילה רביע וחומש ושתות נמצא בס‫' | |style="text-align:right;"|אומר תחילה רביע וחומש ושתות נמצא בס‫' | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Denominator:'''</span> Their sum is 37 and this is the denominator. | |
| + | :<math>\scriptstyle{\color{blue}{\left(\frac{1}{4}\sdot60\right)+\left(\frac{1}{5}\sdot60\right)+\left(\frac{1}{6}\sdot60\right)=15+12+10=37}}</math> | ||
|style="text-align:right;"|וכללם הוא ל"ז והוא המחלק | |style="text-align:right;"|וכללם הוא ל"ז והוא המחלק | ||
|- | |- | ||
| | | | ||
| − | : | + | :If you want to know how many cubits the one who sold [one cubit] for 4 dinar sold: |
| − | + | |style="text-align:right;"|ואם תרצה לדעת כמה אמות מכר אותו שמכר בד' דינרי‫' | |
| − | |style="text-align:right;"|ואם תרצה לדעת כמה אמות מכר אותו שמכר בד' דינרי‫' | ||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | ::the money he received: | + | :<span style=color:green>'''Rule of Three:'''</span> Take the quarter of 60, which is 15. Multiply it by the measure of the cloth, which is 30; say: 15 times 30 is 450. |
| + | |style="text-align:right;"|תפוס הרביע מס' שהוא ט"ו ותרבע אותו על סך הבגד שהוא ל' ואמור ט"ו פעמי' ל' ת"נ | ||
| + | |- | ||
| + | | | ||
| + | :Divide it by 37; the result is 12 and 6 parts of 37. | ||
| + | :<math>\scriptstyle{\color{blue}{a_1=\frac{\left(\frac{1}{4}\sdot60\right)\sdot30}{37}=\frac{15\sdot30}{37}=\frac{450}{37}=12+\frac{6}{37}}}</math> | ||
| + | |style="text-align:right;"|חלקם בל"ז יבא י"ב ו' חלקי' מל"ז | ||
| + | |- | ||
| + | | | ||
| + | :So, he sold 12 cubits and 6 parts of 37. | ||
| + | |style="text-align:right;"|הרי שמכר [הה] י"ב אמות ו' חלקי' מל"ז | ||
| + | |- | ||
| + | | | ||
| + | :If you want to know how much money he received: | ||
|style="text-align:right;"|ואם תרצה לדעת כמה מעות קבל | |style="text-align:right;"|ואם תרצה לדעת כמה מעות קבל | ||
|- | |- | ||
| | | | ||
| − | :::<math>\scriptstyle{\color{blue}{4\sdot12=48}}</math> | + | :First, say: he received 48 dinar for 12 cubits. |
| + | :<math>\scriptstyle{\color{blue}{4\sdot12=48}}</math> | ||
|style="text-align:right;"|אמור תחילה מי"ב אמות קבל מ"ח דינ‫' | |style="text-align:right;"|אמור תחילה מי"ב אמות קבל מ"ח דינ‫' | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> For the 6 parts of 37 do this way: if 37, which is a whole cubit, is worth 48 pešuṭim, how much are 6 parts of 37 worth? | |
| + | :<math>\scriptstyle{\color{blue}{37:48=6:X}}</math> | ||
|style="text-align:right;"|ומן הו' חלקי' מל"ז עשה על זה הדרך אם ל"ז שהוא אמה שלמה שוה מ"ח פשי' הו' חלקי' מל"ז כמה שוים | |style="text-align:right;"|ומן הו' חלקי' מל"ז עשה על זה הדרך אם ל"ז שהוא אמה שלמה שוה מ"ח פשי' הו' חלקי' מל"ז כמה שוים | ||
|- | |- | ||
| | | | ||
| − | :: | + | :Say: 6 times 48 is 288. |
| − | |style="text-align:right;"|אמור ו' פעמי' מ"ח רפ"ח | + | |style="text-align:right;"|אמור ו' פעמי' מ"ח רפ"ח |
| − | |||
|- | |- | ||
| | | | ||
| − | ::the same for the others | + | :Divide it b 37; the result is 7 pešuṭim and 29 parts of 37. |
| + | :<math>\scriptstyle{\color{blue}{X=\frac{6\sdot48}{37}=\frac{288}{37}=7+\frac{29}{37}}}</math> | ||
| + | |style="text-align:right;"|חלקם בל"ז יבא ז' פשי' וכ"ט חלקים מל"ז | ||
| + | |- | ||
| + | | | ||
| + | :Do the same for the others and you will get the answer. | ||
|style="text-align:right;"|וכזה תעשה האחרי' ויצא לך העניין | |style="text-align:right;"|וכזה תעשה האחרי' ויצא לך העניין | ||
|- | |- | ||
| | | | ||
|} | |} | ||
| + | |||
| + | == Apparatus == | ||
| + | |||
| + | <div class="mw-collapsible mw-collapsed"><div class="mw-collapsible-content"> | ||
| + | <references /> | ||
| + | </div></div> | ||
== Appendix: Bibliography == | == Appendix: Bibliography == | ||
| Line 5,652: | Line 7,512: | ||
:4) Moscow, Russian State Library, Ms. Guenzburg 698/2 (IMHM: f 47914), ff. 10r-40v (14th-15th century) | :4) Moscow, Russian State Library, Ms. Guenzburg 698/2 (IMHM: f 47914), ff. 10r-40v (14th-15th century) | ||
:5) Oxford, Bodleian Library MS Mich. 536/6 (IMHM: f 22082), ff. 88r-99v; 110r-117v (cat. Neub. 1268, 6); (1518-1520)<br> | :5) Oxford, Bodleian Library MS Mich. 536/6 (IMHM: f 22082), ff. 88r-99v; 110r-117v (cat. Neub. 1268, 6); (1518-1520)<br> | ||
| + | |||
| + | <span style=color:blue>The transcript is based mainly on manuscript Oxford 536</span> | ||
| + | |||
'''<u>Bibliography</u>:'''<br> | '''<u>Bibliography</u>:'''<br> | ||
Steinschneider, Moritz. 1906. Mathematik bei den Juden, Band II: 1551-1840. Monatsschrift für die Geschichte und Wissenschaft des Judenthums 50, p. 214. repr.: ed. Gad Freudenthal, Hildesheim, Zürich, New York: Olms, 2014, p. 137. | Steinschneider, Moritz. 1906. Mathematik bei den Juden, Band II: 1551-1840. Monatsschrift für die Geschichte und Wissenschaft des Judenthums 50, p. 214. repr.: ed. Gad Freudenthal, Hildesheim, Zürich, New York: Olms, 2014, p. 137. | ||
Latest revision as of 18:43, 22 May 2023
Contents
- 1 Problems of Various Types
- 1.1 Find a Number Problem - Subtraction of Fractions
- 1.2 Find a Number Problem - Addition of Fractions
- 1.3 Find a Quantity Problem - Whole from Parts Problem - Lance
- 1.4 How Much Problem - Money
- 1.5 Find a Quantity Problem - First from Last Problem – Money
- 1.6 How Much Problem – Money
- 1.7 How Much Problem – Money
- 1.8 Pricing Problem - Find the Amount
- 1.9 Pricing Problem - Find the Price
- 1.10 Exchange Problem - Currencies
- 1.11 Motion Problem – Pursuit
- 1.12 Motion Problem – Encounter
- 1.13 How Many Problem - Group of People
- 1.14 Buy and Sell Problem
- 1.15 Boiling Problem
- 1.16 Partnership Problem - For the Same Time
- 1.17 Purchase Problem – Moneychanger
- 1.18 Payment Problem - Digging a Hole
- 1.19 Buy and Sell Problem
- 2 Exchange Problems
- 3 Interest and Pricing Problems
- 4 Problems of Various Types
- 4.1 Rent Problem
- 4.2 Exchange Problems
- 4.3 Proportions of Fractions
- 4.4 Find a Quantity Problem - Whole from Parts – Tree
- 4.5 Motion Problem - To and From - an Ant Climbing a Tower
- 4.6 Find a Quantity Problem - First from Last Problem – Money
- 4.7 Divide a Quantity – Money
- 4.8 Divide a Quantity – Money
- 4.9 Ordering Problem - Six Coins
- 4.10 Find a Number Problem - Ratio of Integers and Fractions
- 4.11 Multiple Quantities - Boys Selling Eggs
- 4.12 Find a Quantity Problem - Whole from Parts – Fish
- 4.13 Simultaneous Division - Money in a Purse
- 4.14 Partnership - for the same time
- 4.15 Give and Take Problem - gatekeeper
- 4.16 Shared Work Problem - Rivers Filling a Fountain
- 4.17 Motion Problem – Pursuit
- 4.18 Mixture and Alligation Problem - Goldsmith making rings from several kinds of coins
- 4.19 Divide a Quantity - Price of a Fish Paid by a Group of People
- 4.20 Joint Purchase Problem - If You Give Me - two men - Amounts of Money
- 4.21 Interest and Discount Problem - find the fund
- 4.22 Exchange Problems
- 4.23 Interest and Discount Problem
- 4.24 Pricing Problem - Find the Amount
- 4.25 Divide a Quantity - Sharing Food
- 4.26 Find a Number Problems - Multiplication of fractions
- 4.27 Multiple Quantities - Coins that Worth an Amount of Money
- 4.28 Find a Number Problems - Sums
- 4.29 Find a Number Problem - Multiplication of integers and fractions
- 4.30 Interest and Discount Problems
- 4.31 Multiple Quantities Problem - Five Weights
- 4.32 Guessing - coins
- 4.33 Payment Problem - three workers, three different daily wages, the same actual payment
- 4.34 Divide a Quantity Problem - Proportional Division – Inheritance
- 5 Geometrical Problems
- 5.1 Triangulation Problem - Two towers
- 5.2 Rectangle
- 5.3 Quadrangle
- 5.4 Triangulation Problem - Tree leaning against a wall
- 5.5 Transformation Problems
- 5.6 Volume
- 5.7 Questions about Rectangles
- 5.8 Square
- 5.9 Area
- 5.10 Equilateral Triangle
- 5.11 Scalene Triangle
- 5.12 Isosceles Triangle
- 5.13 Area
- 5.14 Divide a Figure
- 5.15 Rhombus
- 5.16 Area
- 6 Additional Problems
- 7 Apparatus
- 8 Appendix: Bibliography
Problems of Various Types |
|||||||||||
| [Gold Weights: 1 kikkar = 100 liṭra; 1 liṭra = 20 dinar/zuz; 1 dinar/zuz = 12 pešiṭim/pešuṭim] | |||||||||||
Find a Number Problem - Subtraction of Fractions |
|||||||||||
|
|
|||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
|
[1]א אם ישאלך אדם כמה הוא יותר השליש מן הרביע | ||||||||||
|
אמור ג' פעמים ד' הם י"ב | ||||||||||
|
השליש הוא ד' דנריצימו | ||||||||||
|
הרביע הוא ג' דנריציסמו | ||||||||||
|
נמצא שהשליש הוא יותר מן הרביע א' תריציסמו | ||||||||||
|
וכן אם ישאלך כמה יותר הרביע מן החומש | ||||||||||
|
תעשה כמו שעשינו למעלה ואמור ד' פעמים ה' הם כ' | ||||||||||
|
הרביע הוא ה' בינטיני | ||||||||||
|
החומש הוא ד' בינטיני | ||||||||||
|
נמצא שהרביע הוא יותר מן החומש א' בינטינו | ||||||||||
| Likewise for everything similar to it. | וכן כל הדומה לו | ||||||||||
|
וכן אם ישאלך כמה הוא יותר השליש מן החמישית | ||||||||||
|
אמור ג' פעמים ה' הם ט"ו | ||||||||||
|
השליש הוא ה' קוויניציסמי | ||||||||||
|
והחומש הוא ג' קוויניציס | ||||||||||
|
נמצא שהשליש הוא יותר מן החומש ב' קוויניציסמו | ||||||||||
Find a Number Problem - Addition of Fractions |
|||||||||||
|
ב אם ישאלך אדם כמה הוא שליש פשוט ורביע וחומש ושתות ושביעית | ||||||||||
|
תצטרך לחלק הפשוט לכל כך חלקי' שתמצא בו כל אלו השעורים | ||||||||||
|
וכלך לדרך זו ואמור ג' פעמים ד' הם י"ב ה' פעמין י"ב הם ס' | ||||||||||
|
| |||||||||||
|
השליש הוא תת"מ חלקי' | ||||||||||
|
הרביע הוא תר"ל חלקי' | ||||||||||
|
החומש הוא תק"ד חלקי' | ||||||||||
|
השתות הוא ת"כ חלקי' | ||||||||||
|
השבעית הוא ש"ס חלקי' | ||||||||||
|
הרי שמן כולם הם אלפי' ותשנ"ד חלקי' | ||||||||||
|
תוציא מהם אלפים ותק"כ חלקי' שהוא אחד שלם נשארו בידך רל"ד חלקי' | ||||||||||
|
נמצא ששליש פשוט ורבע וחומש ושתות ושביעי הוא א' פשוט שלם ורל"ד חלקי' מפשוט אחד שתחלקהו לאלפי' ותק"כ חלקי' שהוא קי"ז חלקי' מאלף ור"ס וכה"ל | ||||||||||
|
| |||||||||||
Find a Quantity Problem - Whole from Parts Problem - Lance |
|||||||||||
|
ג שאלה רומח אחד שלישיתו במים ורביעיתו בעפר ולמעלה מן המים י' אמות כמה אמות כל הרומח | ||||||||||
|
נבקש מניין שיש לו שלישית ורביעית והוא י"ב | ||||||||||
|
ושלישיתו ורביעיתו מחוברים ז' | ||||||||||
|
נחסרם מי"ב ישארו ה' | ||||||||||
|
כפלנו הקצוות עלו ק"כ | ||||||||||
|
חלקנום על ה' עלה כ"ד וזהו גבהות כל הרומח | ||||||||||
|
שלישיתו ח' ורביעיתו ו' נחסרם מכ"ד ישארו י' שלמים בלי תוספת ומגרעת וכה"ל | ||||||||||
How Much Problem - Money |
|||||||||||
|
ד שאלה ממון חברנו חמישיתו ושביעיתו ותשיעיתו והיו י' כמה הממון | ||||||||||
|
נבקש המורה והוא שט"ו והחלקי' ההם קמ"ג | ||||||||||
|
כאשר החלק שט"ו של ה' יעלו ס"ג | ||||||||||
|
וכשהחלק של ז' יעלו מ"ה | ||||||||||
|
וכשהחלק של ט' יעלו ל"ה | ||||||||||
|
חברם יחד יעלו קמ"ג | ||||||||||
|
נכפול שט"ו על י' עלו ג' אלפי' וק"נ | ||||||||||
|
חלקנום על קמ"ג עלו כ"ב שלמי' וד' חלקי' מן קמ"ג וככה הממון | ||||||||||
|
קח חמישית כ"ב הם ד' נשאר ב' שהם ב' פעמי' קמ"ג והם רפ"ו | ||||||||||
|
חבר עליהם ד' חלקי' הרי ר"צ | ||||||||||
|
חלקם בה' יעלו נ"ח חלקי' מקמ"ג | ||||||||||
|
|
|||||||||||
|
וכן עשה מן התשיעית והשבעית ויעלו י' | ||||||||||
|
|
|||||||||||
|
עוד יש בו כלל אחר | ||||||||||
|
שתאמר החלקי' שהוספת שהם י' הם קמ"ג והממון הראשון היה קע"ב | ||||||||||
|
אמור אם קמ"ג שוים י' קע"ב כמה שוים | ||||||||||
|
אמור י' פעמי' קע"ב הם אלף תש"כ חלקם על קמ"ג יבאו י"ב שלמי' וד' חלקי' מקמ"ג | ||||||||||
|
חבר אליהם הי' יהיו כ"ב שלמי' וד' חלקי' מקמ"ג בלי תוספת ומגרעת | ||||||||||
|
וכן כ.. מה הכלל ג"כ הוא טוב לדעת מדת הרומח הכתוב למעלה | ||||||||||
Find a Quantity Problem - First from Last Problem – Money |
|||||||||||
|
[2]ה נעשה להפך ממון חסרנו ממנו חמשיתו ושבעיתו ותשיעיתו ונשארו י' | ||||||||||
|
נחסר קמ"ג שהם השברי' משט"ו שהוא המורה ישאר קע"ב | ||||||||||
|
ונעשה כך כפלנו י' על שט"ו עלו ג' אלפי' וק"נ חלקנום על קע"ב עלו י"ח שלמי' ונ"ד חלקי' מקע"ב | ||||||||||
|
לקחנו חמשית ושבעית ותשעית של זה המספר ישאר י' שלמי' | ||||||||||
|
| |||||||||||
How Much Problem – Money |
|||||||||||
|
ו שאלה ממון הוספנו עליו מחציתו ושלישיתו וחמישיתו וששיתו ובסך הכל היה מ' כמה היה הממון | ||||||||||
|
ידענו כי החצי והשלישית והששית הוא אחד שלם ונחשוב כי היה אלו אחד הרי שנים יש לו תוספת החמישית | ||||||||||
|
| |||||||||||
|
עתה יש לנו לחלק מ' על ב' וחמישית והעולה הוא הממון הנה נקח לכל אחד מן השלמים ה' ונשים עמהם החמשית יהיו אלף | ||||||||||
How Much Problem – Money |
|||||||||||
|
ז שאלה לקחנו חמישית ממון גם שביעיתו ותשיעיתו כמה הוא מערך הממון | ||||||||||
|
נבקש המורה והוא שט"ו | ||||||||||
|
ונחבר חמישית ושביעית ותשיעית יהיו קמ"ג | ||||||||||
|
נחלקה על ל"ה והנה הם ד' תשעיות ונשארו ג' חלקי' מל"ה כי ל"ה הוא התשיעית וה' הוא שביעית התשיעית | ||||||||||
|
| |||||||||||
Pricing Problem - Find the Amount |
|||||||||||
|
ח שאלה אדם מוכר י"ג מדות בכ"ג כמה מדות יתן בז' פשוטי' | ||||||||||
|
נאמר ז' פעמי' י"ג יהיו צ"א נחלקם על כ"ג בא ג' מדות וכ"ב מכ"ג חלקי' במדה אחת | ||||||||||
Pricing Problem - Find the Price |
|||||||||||
|
ועת' נהפוך חשבון שנבקש לדעת בכמה יתן לו ז' מדות | ||||||||||
|
נאמר ז' פעמים כ"ג הם קס"א נחלקם על י"ז יהיו י"ב וה' חלקי' מי"ג בפשוט | ||||||||||
Exchange Problem - Currencies |
|||||||||||
|
וכן אם יאמר לך אדם אם ג' תרוביסים שוים ד' קורטונוס ה' תרוביסיט' כמה קורטונוס שוים | ||||||||||
|
אמור ה' פעמי' ד' הם כ' חלקם על ג' בא ו' קורטוניס וב' שלשי' וכה"ל | ||||||||||
Motion Problem – Pursuit |
|||||||||||
|
ט שאלה אדם שלח רץ שילך בכל יום כ"ט מילים אחר מהלך י' ימי' שלח רץ אחר אחריו שילך בכל יום ל"ז מילי' | ||||||||||
|
נכפול המילים שהלך בי' ימי' יהיו ר"צ נחלקם על היתרון שבין שני המהלכים שהוא ח' והנו ל"ו ימים ורבע יום | ||||||||||
Motion Problem – Encounter |
|||||||||||
|
י שאלה ראובן יצא מעירו ללכת לקראת שמעון לעירו בקר יום ראשון של ר"ח ובאותו יום עצמו יצא שמעון מעירו ללכת לעיר ראובן | ||||||||||
|
ככה תעשה חבר שני המהלכי' הם ל"ו חלק עליו הק' מילים יהיו ב' ימים ישארו כ"ח חלקי' מל"ו ביום אחד שהם ז' תשעיו' יום | ||||||||||
How Many Problem - Group of People |
|||||||||||
|
יא שאלה אדם עבר על אנשים אמר להם שלו' לכם מאה איש | ||||||||||
|
והנה נקח למספרם אחד ואחד כמונו והנה שנים ומחציתנו חצי אחד הנה שנים וחצי נוסיף רבעיתו יהיו [3]ג' רבעיות | ||||||||||
|
ובעבור שיש לנו רבעית נקח לכל שלם ד' ויהיו ח' נחבר אליהם הג' רבעים יהיו י"א | ||||||||||
|
| |||||||||||
|
ובעבור שאמרו שיהיו עמו מאה יהיה מספרם עם התוספת צ"ט | ||||||||||
|
נשיבם מהדרך הד' יהיו שצ"ו | ||||||||||
|
נחלקם על י"א יהיו ל"ו וככה מספרם | ||||||||||
Buy and Sell Problem |
|||||||||||
|
יב שאלה אדם קנה בק' זהובים ק' ליט' אחרי כן מכר הנ' ליט' ורבע ליט' בזהוב והנ' ליט' מכר ליט' פחות רבע ליט' בזהוב | ||||||||||
|
נשיב הנ' ראשונים ר' כי רבעים הם נחלקם על ה' כי ליט' ורבע ליט' מכר בזהוב יהיו מ' זהובי' | ||||||||||
|
גם נכפול הנ' אחרים על ד' יהיו ר' נחלקם על ג' כי ג' רבעים מכר בזהוב והנה יהיו ס"ו זהובים ושני שלישי זהוב | ||||||||||
|
וחבר אליהם המ' יהיה הריוח ו' זהובים ושני שלישי זהוב וכה"ל | ||||||||||
|
| |||||||||||
Boiling Problem |
|||||||||||
|
יג שאלה אדם היו לו י' מדות משמש ורוצה לבשלם עד שלא ישאר כי אם השלישית והנה החל לבשל עד שנשארו מהם ח' מדות | ||||||||||
|
ועתה יש לך ג' מספרים ידועים | ||||||||||
|
האחד שליש י' והוא ג' ושליש | ||||||||||
|
והשני ח' הם המדות שנתבשלו | ||||||||||
|
והשלישי ו' שנשארו מן הנשפך | ||||||||||
|
והנה נכפול ו' על ג' ושליש יהיו כ' וחלקם על ח' יהיו ב' וחצי | ||||||||||
Partnership Problem - For the Same Time |
|||||||||||
|
יד שאלה ד' אנשים יש לאחד מהם י"א דינרי' ולשני י"ג די' ולשלישי ט"ו דינרי' ולרבעי י"ז דינרי' והרויחו י"ט דינרי' | ||||||||||
|
נחבר ראשי' כל ד' ממונם ויהיו נ"ו | ||||||||||
|
ובערך כל אחד אל נ"ו ככה יקח מי"ט | ||||||||||
|
ונעשה כך נכפול י"א על י"ט יעלו ר"ט נחלק על נ"ו יעלו ג' שלמי' ומ"א חלקי' | ||||||||||
|
עשינו כן בי"ג עלו רמ"ז חלקנום על נ"ו עלו ד' שלמי' וכ"ג חלקי' | ||||||||||
|
עשינו כן בט"ו יהיו רפ"ה חלקנום על נ"ו עלו ה' שלמי' וה' חלקי' | ||||||||||
|
עשינו כן בי"ז עלו שכ"ג חלקנום על נ"ו עלו ה' שלמי' ומ"ג חלקי' | ||||||||||
|
חברנו אלו השלמי' ואלו החלקי' עלו י"ט שלמי' כי החלקי' האלו חלקי נ"ו הם | ||||||||||
Purchase Problem – Moneychanger |
|||||||||||
|
טו שאלה יש אצל המחליף ג' מטבעים והזהוב שוה ממטבע אחד ג' דינרי ומן השני ד' ומן השלישי ו' ובא אדם אחד ובקש למחליף שיתן לו מג' המטבעים בזהוב ויהיה המספר שוה מן היקרים כמו משאינם יקרים | ||||||||||
|
בקש היתרה שיהיה בו שלישית ורבעית וששית והוא י"ב | ||||||||||
|
החלקי' הם ט' והוא דינר | ||||||||||
|
נחלק המורה על זה המספר יהיו י"ב תשי' וככה לקח מכל מטבע | ||||||||||
|
אחר על זאת השאלה שתאמר מה ערך י"ב אל ט' והיה כמות שלישיתו והנה נוסיף על י"ב ד' יהיו י"ו | ||||||||||
Payment Problem - Digging a Hole |
|||||||||||
|
יו שאלה ראובן שכר שמעון שיחפור לו בקרקע ז' באורך וו' ברוחב וה' בעומק ויתן לו י"א פשיטי' והוא חפר ו' באורך ה' ברוחב ד' בעומק | ||||||||||
|
נעשה כך ז' פעמים ו' הם מ"ב כפלם על ה' שהוא העומק ויהיו ר"י | ||||||||||
|
גם נכפול המספר השני שהוא ו' על ה' והם ל' גם נכפול זה על ד' שהוא העומק יהיו ק"כ | ||||||||||
|
ונכפול י"א פעמי' [4]ק"כ הם אלף וש"כ חלקם על ר"י עלו ו' שלמי' ונשאר ס' שהם ב' שבעיות פשוט וכה"ל | ||||||||||
Buy and Sell Problem |
|||||||||||
|
יז שאלה אדם קנה ג' חמשיות ליט' בפשוט ומכר ד' שבעיות ליט' בפשוט והרויח פשוט כמה היה ממונו | ||||||||||
|
בקש המורה הוא ל"ה וכפול ה' על ז' | ||||||||||
|
והיה ג' חמשיותיו כ"א | ||||||||||
|
וד' שבעיותיו כ' והממון היה כ' וכה"ל | ||||||||||
|
נראה שרוצה לו' שהוצ' כ' פשוטי' וזהו | ||||||||||
|
יח שאלה אדם קנה ד' שבעיות ליט' בפשוט ומכר ה' תשעיות ליט' בפשוט והרויח פשוט כמה היה הממון | ||||||||||
|
ידוע הוא כי ד' שבעיות אחד הוא יותר מה' תשעיות אחד | ||||||||||
|
והנה המורה ס"ג | ||||||||||
|
וה' תשיעיותיו ל"ה | ||||||||||
|
וד' שבעיותיו ל"ו | ||||||||||
|
ותוכל לבחון זה כי אחר שקנה ד' שבעיות ליט' בפשוט ממונו ל"ה הנה יש לו כ' ליט' | ||||||||||
|
עשה מהם תשעיו' יהיו ק"פ | ||||||||||
|
חלק זה המספר על ה' כי ה' תשעיו' מכר בפשוט יעלו בידך ל"ו | ||||||||||
|
ואילו אמר כי הרויח ב"פ | ||||||||||
|
כפלם על ל"ה יהיו הפשוטי' שהוציא | ||||||||||
|
|
כי הממון יהיה מ' ליט' | ||||||||||
|
ואם אמר ג"פ | ||||||||||
|
יכפלם על ל"ה | ||||||||||
|
וככה עד סוף החשבון | ||||||||||
Exchange Problems |
|||||||||||
|
יט כלל כל החשבונות הוא על שלשה פנים במדה או במשקל או במספר | ||||||||||
|
על כן נאמר הריגולי' הנאותים לשלשתם | ||||||||||
|
אם ישאל לך שום חשבון שיהיה בו אחד מאלו הג' מינים נרבה אותו הדבר שנרצה לידע כנגד אותה שאיננה ממינה עצמה ממש | ||||||||||
|
המשל בזה אם ז' פיסט' שוים ט' קורטוניס כמה ישוו ק' ליט' מפיסני' לקורטו' | ||||||||||
|
כבר ידעת כי הקורטו' הוא הדבר שאינה ממינה עצמה ממש לכן נרבה ט' פעמי' ק' יהיו תת"ק | ||||||||||
|
וחלקם על ז' קכ"ח ליט' וי"א דינרי' והם וכך ישוו הק' ליט' מפיסני' לקורטו' | ||||||||||
|
ודע כי אם ז' פיסני' שוים ט' קורטו' הז' דינרי' פיסני' שוים ט' דינרי' קורטוני' והז' ליט' מפיסני' שוים ט' ליט' קורטו' ות"ש ליט' מפיסני' שוים תת"ק ליט' קורטו' | ||||||||||
| ? | וכן יבא בין פפריני' ופריביסיני' ולכל [חשבון] כזה | ||||||||||
|
כ אם ישאל לך חשבון שיהיה בו מאלו הג' מינים ולפני הב' חלקי' יהיו שבורים | ||||||||||
|
נדע באיזה המספר ימצאו אלו השבורים | ||||||||||
|
ואחר שידעת באי זה המספר ימצאו אלו השבורים נרבה הב' חלקי' באותו המספר שימצאו בו אלו השבורים | ||||||||||
|
אחרי כן נרבה אותו הדבר שנרצה לדעת כנגד אותו הדבר שאנינה ממינה עצמה ונחלק אותה על השלישית וכה"ל | ||||||||||
|
| |||||||||||
|
כא ואם נשאל לך חשבון אחר שיהיה שבור מחלק אחד | ||||||||||
|
נרבה הב' חלקי' באותו המספר דהיינו עם אותו השבור | ||||||||||
|
אחרי כן נרבה אותו הדבר שנרצה לידע כנגד אותו הדבר שאנינה ממינה ונחלק אותה על השלישית | ||||||||||
|
| |||||||||||
| We give an example for the two cases - one for a calculation in which one part involves a fraction and the other in which the two parts involve fractions: | ונשים המשל בשני פנים האחד בעבור החשבון שהוא שבור מחלק אחד והאחר שהוא שבור מב' חלקי' | ||||||||||
|
אם ה' פיסני' ורביע שוים ז' קורטונו' כמה ישוו אלף ליט' מפיסני' | ||||||||||
|
וזה החשבון הוא שבור מחלק אחד וזה השיבור הוא הרביע לכן נרבה הב' חלקי' עם ד' | ||||||||||
|
ואמור ד' פעמי' ה' פיסני' ורביע יבוא כ"א פיסני' | ||||||||||
|
וד' פעמי' ז' קורטו' בא כ"ח קורטו' | ||||||||||
|
הרי שכ"א פיסני' שוים כ"ח קורטו' | ||||||||||
|
ונחלק לשביע בעבור [5]שבשני החלקי' ימצא שביע | ||||||||||
|
השביע מכ"א הוא ג' | ||||||||||
|
השביע מכ"ח הוא ד' | ||||||||||
|
נמצא שג' פיסני' שוים ד' קורטו' | ||||||||||
|
ואנחנו נרצה לידע כמה ישוו אלף ליט' מפיסני' הפיסני הוא הדבר שנרצה לידע והקורטו' הוא הדבר שאנינה ממינה עצמה | ||||||||||
|
לכן נרבה ד' פעמים אלף יבא ד' אלפי' מקורטו' | ||||||||||
|
ונחלק בג' שיבא אלף ושל"ג ליט' וו' דינרי' וח"פ וכן ישוו אלף ליט' מפיסני לקורטוט' | ||||||||||
| We give an example of a calculation in which both parts involve fractions: | ונשים המשל אל החשבון שהוא שבור מב' חלקיו | ||||||||||
|
אם ז' אונקיו' ושליש כסף שוים ע"ג ליט' וה' דינרי' מפיסני' כמה ישוו הי"ט אונקיו' כסף לפי זה החשבון | ||||||||||
|
ואלו השבורים הם שליש ורביע אנה ימצאו בי"ב | ||||||||||
|
לכן יש לנו לרבות הב' חלקי' בי"ב | ||||||||||
|
ואמור י"ב פעמי' ז' אונקיו' ושליש יבא פ"ח אונקיו' כסף | ||||||||||
|
וי"ב פעמי' ע"ג ליט' וה' דינרי' יהיו תתע"ט ליט' מפסני' | ||||||||||
|
הרי שפ"ח אונקיות כסף שוים תתע"ט ליט' | ||||||||||
|
ונרצה לידע כמה ישוו הי"ט אונקיו' כסף | ||||||||||
|
נרבה י"ט פעמי' תתע"ט ליט' יהיו י"ו אלפי' ותש"א | ||||||||||
|
חלקם בפ"ח יבא קפ"ט ליט' וט"ו די' וח"פ וב' חלקי' מי"א וכן ישוו הי"ט אונקיו' כסף באותו החשבון | ||||||||||
Interest and Pricing Problems |
|||||||||||
| Find the Time | |||||||||||
|
כב שאלה הככר ירויח השנה ל"ו ליט' וי"ח די' נוסף הס"ה ליט' בכמה זמן ירויחו כהם | ||||||||||
|
תרבה הק' ליט' כנגד י"ב חדשי השנה ואמור י"ב פעמי' ק' חדשים הם אלף ור' | ||||||||||
|
חלקם על ס"ה יבא לכל חלק י"ח חדשי' וי"ג ימים וי"א חלקי' מי"ג חלקי' ביום | ||||||||||
|
נמצא שבי"ח חדשי' וי"ג ימי' וי"א חלקי' מי"ג ביום ירויחו ס"ה ליט' כלכך כמו שירויח הככר השנה | ||||||||||
| Find the Earned Interest | |||||||||||
|
כג שאלה אם יאמר אדם הלותי מעות לחשבון גפה"ח הליט' החדש כמה יבואו הס' ליט' ח' חדשים | ||||||||||
|
תרבה מעות הרבית עם החדשים ואמור ג' פעמי' ח' כ"ד שהם ב' דינרי' | ||||||||||
|
אחר כן תרבה אלו הב' די' עם הס' ליט' ואמור ס' פעמי' ב' דינרי' הם ק"כ די' שהם ו' ליט' | ||||||||||
|
נמצא שבס' ליט' לחשבון גפה"ח יבוא ו' ליט' לח' חדשים וכה"ל | ||||||||||
| Find the Fund | |||||||||||
|
כד אם ישאלך אדם הלותי מעות הליט' לחשבון כך וכך החדש כמה ליט' ירויחו היום א"פ | ||||||||||
|
נחלק ל' ליט' בכל כך חלקי' כמו המעות שירויח הליט' החדש | ||||||||||
|
המשל בזה הרי שהלותי הליט' בב' פשוטי' וחצי החדש ותרצה לידע כמה ליט' ירויחו היום א"פ | ||||||||||
|
נחלק ל' ליט' בב' וחצי שיבוא י"ב ליט' | ||||||||||
|
נמצא שבי"ב ליט' ירויחו היום א"פ וכה"ל | ||||||||||
| Find the Time | |||||||||||
|
כה אם ישאלך אדם הלותי הליט' לחשבון כך וכך החודש בכמה ימים ירויח הליט' א"פ | ||||||||||
|
חלק ל' יום בכל כך חלקי' כמו המעות שירויח הליט' החדש | ||||||||||
|
המשל בזה הרי שהלוית לחשבון ג' ותרצה לידע כמה ימים ירויח הליט' א"פ | ||||||||||
|
חלק ל' יום בג' חלקי' שיבוא י' ימים | ||||||||||
|
הרי שבי' ימים ירויח הליט' א"פ | ||||||||||
| Find the Fund | |||||||||||
|
כו אם ישאלך אדם הככר ירויח כל השנה כל כך ליט' כמה ליט' ירויחו היום א"פ | ||||||||||
|
חלק ק"נ ליט' בכל כך חלקי' כמו הליט' שירויח הככר השנה | ||||||||||
|
המשל בזה הככר ירויח י"ב ליט' השנה ותרצה לידע כמה ליט' ירויחו היום א"פ | ||||||||||
|
חלק ק"נ ליט' בי"ב חלקי' שיבוא [6]י"ב וחצי | ||||||||||
|
הרי שבי"ב ליט' וחצי ירויחו ביום א"פ וכה"ל | ||||||||||
| Find the Time | |||||||||||
|
כז אם ישאלך אדם הככר ירויח כל כך ליט' השנה בכמה ימים ירויח הליט' א"פ | ||||||||||
|
חלק ק"נ ימים בכל כך חלקי' כמו הליט' שירויח הככר השנה | ||||||||||
|
המשל בזה הככר ירויח ט' ליט' השנה ותרצה לידע בכמה ימים ירויח הליט' א"פ | ||||||||||
|
חלק ק"נ ימים בט' חלקי' שיבואו י"ו ימים וב' שלשי הרי שבי"ו ימים וב' שלישי ירויח הליט' א"פ וכה"ל | ||||||||||
|
כח אם ישאלך אדם כל כך ליט' ירויחו כל כך בכל כך זמן | ||||||||||
|
תרבה סכום הליט' כנגד סכום החדשים וחלק בכל כך חלקי' כמו שהם ליט' או חדשים | ||||||||||
|
המשל בזה נ' ליט' ירויחו ג' ליט' בד' חדשים הע"ה ליט' בכמה זמן ירויחו כהם | ||||||||||
|
הרבה סכום הליט' כנגד סכום החדשים ואמור נ' פעמי' ד' חדשים יבא ר' חדשים וחלקם בע"ה יבא ב' חדשים וכ' ימים נמצא שבב' חדשי' וב' ימים ירויחו הע"ה ליט' כל כך כמו הנ' ליט' ד' חדשים וכה"ל | ||||||||||
| Find the Fund | |||||||||||
|
כט אם ישאלך אדם כל כך ליט' ירויחו בכל כך זמן כל כך ליט' כמה ליט' בכל כך זמן ירויחו כהם | ||||||||||
|
תרבה סכום החדשים כנגד סכום הליט' וחלקם בכל כך חלקי' כמו החדשי' או הליט' שנרצה לידע | ||||||||||
|
המשל בזה כ"ה ליט' ירויחו בו' חדשי' מ' דינרי' כמה ליט' ירויחו בהם בח' חדשים | ||||||||||
|
תרבה סכום החדשים כנגד סכום הליט' ואמור ו' פעמי' כ"ה ליט' יבא ק"נ ליט' חלקם בח' חלקי' יבא י"ח ליט' וט"ו דינרי' | ||||||||||
| Find the Time | |||||||||||
|
ל אם ישאלך אדם הלותי הליט' בכך וכך החדש כל כך ליט' בכמה זמן יכפלו בלתי לשים ריוח אקרן | ||||||||||
|
חלק כ' שנים בכל כך חלקי' כמו המעות שירויח הליט' החדש | ||||||||||
|
המשל בזה הלותי הליט' בגפ"ה הצ"ה ליט' בכמה זמן יכפלו | ||||||||||
|
חלק כ' שנים בג' חלקי' שיבא ו' שנים וח' חדשי' נמצא שבו' שנים וח' חדשים יכפלו הצ"ה ליט' | ||||||||||
|
לא אם ישאלך אדם הככר ירויח השנה כל כך ליט'
כל כך ליט' בכמה זמן יכפלו בלתי לשים ריוח אקרן | ||||||||||
|
חלק ק' שנים בכל כך חלקי' כמו הליט' שירויח הככר | ||||||||||
|
המשל בזה הככר ירויח ח' ליט' השנה המ"ה ליט' בכמה זמן יכפלו | ||||||||||
|
חלק ק' שנים בח' חלקי' שיבא י"ב שנים וחצי נמצא שבי"ב שנים וחצי יכפלו המ"ה ליט' | ||||||||||
| Pricing Problem - Find the Price | |||||||||||
|
לב אם ישאלך אדם הקנטרו' מן המוך שוה י"ז ליט' וה' דינרי' כמה ישוה הליט' | ||||||||||
|
חלק אלו הי"ז ליט' וה' דינרי' בג' חלקי' שיבא לכל חלק ה' ליט' וט"ו די' | ||||||||||
|
אחרי כן חלק אלו הה' ליט' וט"ו די' בה' חלקי' שיבא לכל חלק כ"ג דינרי' | ||||||||||
|
עת' תחלק אלו הכ"ג דינרי' בי"א חלקי' שיבא לכל חלק בה"פ וא' חלק מי"א נמצא שהליט' מן המוך שוה בה"פ וא' חלק מי"א בפשוט | ||||||||||
| Find the Earned Interest | |||||||||||
|
[7]לג אם ישאלך אדם כל כך ליט' ירויחו היום א"פ לאי זה חשבון יבא החדש הליט' | ||||||||||
|
חלק ליט' בכל כך חלקי' כמו הליט' שירויחו היום א"פ | ||||||||||
|
המשל בזה י"ב ליט' ירויחו היום א"פ ותרצה לידע לאי זה חשבון יבא החדש הליט' | ||||||||||
|
חלק ל"פ בי"ב חלקי' שיבא ב"פ וחצי נמצא שיבא החדש הליט' ב"פ וחצי וכה"ל | ||||||||||
|
לד אם ישאלך אדם כל כך ליט' ירויחו היום א"פ כמה ליט' ירויחו ק' ליט' א' שנה | ||||||||||
|
חלק ק"נ ליט' בכל כך חלקי' כמו הליט' שירויחו היום א"פ | ||||||||||
|
המשל בזה ט"ו ליט' ירויחו היום א"פ כמה ירויחו ק' ליט' א' שנה' | ||||||||||
|
חלק ק"נ ליט' בט"ו חלקי' שיבא י' ליט' נמצא שק' ליט' ירויחו א' שנה י' ליט' | ||||||||||
|
לה) אם ישאלך אדם הככר ירויח השנה כ"ט ליט' וי"ג דינרי' וז"פ כמה ליט' ירויחו בהם ז' חדשי' | ||||||||||
|
תרבה חדשי השנה עם סכום הליט' דהיינו ק' ליט' וחלקם בכל כך חלקי' כמו החדשי' שנרצה לידע דהיינו הז' חדשים | ||||||||||
|
ואמור י"ב פעמי' ק' ליט' יבא אלף ור' | ||||||||||
|
חלקם על ז' שיבא קע"א ליט' וח' דינרי' וחצי וו' חלקי' מז' בפשוט | ||||||||||
|
נמצא שקע"א ליט' וח' די' וחצי וו' חלקי' מז' בפשוט ירויחו כ"כ בז' חדשי' כמו הככר א' שנה וכה"ל | ||||||||||
| Find the Time | |||||||||||
|
לו אי"א הככר ירויח כ"כ ליט' השנה בכמה זמן יכפלו בלתי לשים ריוח אקרן | ||||||||||
|
חלק ק' שנים בכל כך חלקי' כמו הליט' שירויח הככר השנה | ||||||||||
|
המשל בזה הככר ירויח י"ד ליט' השנה ותרצה לידע בכמה זמן יכפלו | ||||||||||
|
חלק ק' שנים בי"ד חלקי' שיבא ז' שנים וא' חדש וכ"א ימים וג' חלקי' מז' ביום נמצא שבז' שנים וא' חדש וכ"א ימים וג' חלקי' מז' ביום יכפלו | ||||||||||
|
לז אי"א הלויתי הליט' לחשבון ב"פ וחצי החדש בכמה זמן יכפלו בלתי לשים רבית אקרן | ||||||||||
|
חלק כ' שנים בכל כך חלקים כמו המעות שירויח הליט' החדש | ||||||||||
|
המ"ב הלותי כ' דינרי' לחשבון ב"פ וחצי החדש הליט' ותרצה לידע בכמה זמן יכפלו | ||||||||||
|
חלק כ' שני' בב' וחצי שיבא ח' שני' נמצא שבח' שנים יכפלו וכה"ל | ||||||||||
| Find the Earned Interest | |||||||||||
|
לח אם ישאלך אדם הככר ירויח כ"כ ליט' השנה כמה ליט' ירויחו היום א"פ | ||||||||||
|
חלק ק"נ ליט' בכל כך חלקי' | ||||||||||
|
כמו הליט' שירויחו הככר השנה הב' הככר ירויח י"ג ליט' השנה ותרצה לידע כמה ליט' ירויחו היום א"פ | ||||||||||
|
חלק ק"נ ליט' בי"ג חלקי' שיבא י"א ליט' וי' די' וט"פ וג' חלקי' מי"ג בפשוט וכל כך מעות ירויחו היום א"פ | ||||||||||
|
לט אי"א הלותי הליט' החדש לחשבון כך וכך כמה ירויחו היום ק' ליט' | ||||||||||
|
תרבה ג' ושליש כנגד מעות הרבית | ||||||||||
|
המשל בזה הלותי הליט' לחשבון ג"פ החדש ותרצה לידע כמה ירויחו היום ק' ליט' | ||||||||||
|
תרבה ג' ושליש עם ג' ואמור ג' פעמי' ושליש ג' י"פ נמצא שק' ליט' ירויחו היום י"פ וכה"ל | ||||||||||
|
מ אם ישאלך אדם הככר ירויח כ"כ פשוטי' היום כמה ירויח הככר השנה | ||||||||||
|
תרבה א' וחצי [8]עם המעות שירויח הככר היום | ||||||||||
|
המשל בזה הככר ירויח היום י"פ ותרצה לידע כמה יבוא השנה | ||||||||||
|
תרבה א' וחצי עם י' ואמור א' וחצי פעמי' י' ליט' יבא ט"ו ליט' נמצא שירויח הככר ט"ו ליט' השנה | ||||||||||
|
מא אם ישאלך אדם הככר ירויח היום כ"כ פשוטי' כמה יבא החדש הליט' | ||||||||||
|
חלק בג' ושליש המעות שירויח הככר היום | ||||||||||
|
המשל בזה הככר ירויח היום י"פ ותרצה לידע כמה יבא החדש הליט' | ||||||||||
|
חלק י"פ בג' ושליש יבא ג"פ נמצא שיבא לחשבון גפה"ה וכה"ל | ||||||||||
|
מב אי"א הככר ירויח כל כך ליט' השנה כמה יבא החדש הליט' | ||||||||||
|
חלק לחומש הליט' שירויח הככר השנה ותחשבם פשוט' | ||||||||||
|
המשל בזה הככר ירויח י"ב ליט' השנה ותרצה לידע כמה יבא החדש הליט' | ||||||||||
|
חלק י"ב ליט' | ||||||||||
|
נמצא שיבא החדש הליט' ב"פ וב' חמשי' וכה"ל | ||||||||||
|
מג אם תלוה ק' ליט' א' חדש לחשבון ח' ליט' השנה ותרצה לידע כמה יבא בדרך קצרה | ||||||||||
|
כלך לדרך זו ואמור אם היית מלוה אותם א' חדש לחשבון ח' ליט' השנה היה עולה הריוח ק' פשוטי' שהם ח' די' וד"פ | ||||||||||
|
שיבא לכל ליט' א"פ בחדש | ||||||||||
|
וכשאתה מוסיף עוד ח' ליט' הרי שעולה הריוח בכל ליט' בא' חדש א' פשוט וג' חמשי פשוט | ||||||||||
|
שיבא הככר ק' פשוטי' וס' חומשי פשוט שהוא סך הכל י"ג די' וד"פ וכך יבא הככר החדש | ||||||||||
|
| |||||||||||
|
ולחש' ז' ליט' השנה | ||||||||||
|
יבא לכל ליט' א"פ וב' חומשי פשוט בחדש | ||||||||||
|
שיבא הככר בחדש י"ב די' פד"פ פחות ד' פשוטי' וכה"ל | ||||||||||
|
אחר על זאת השאלה הכתובה למעלה | ||||||||||
|
אם תלוה ק' ליט' א' חדש לחשבון ח' ליט' השנה ותרצה לידע כמה יבא בדרך קצרה | ||||||||||
|
כלך לדרך זו ואמור אם היית מלוה ק' ליט' א' חדש לחשבון כ' די' השנה | ||||||||||
|
יבא החדש כ"פ שהם ה' ידות שלישיון דינר | ||||||||||
|
ואמור ה' פעמי' ח' ידות ח' ליט' הם מ' ידות שלישיון שהם י"ג די' וד"פ | ||||||||||
|
ולחשבון זה תאמ' ג"כ ה' פעמי' ז' ידות הם ל"ה ידות שהם י"ב די' פחות ד"פ וכה"ל | ||||||||||
| Find the Interest | |||||||||||
|
מד) אם ישאלך אדם הלותי כ' די' שנה אחת לחש' דפ"ה ובסוף השנה פרעתי מהם קצת הלותים שנה שניה לחשבון דפ"ה כמו כן ופרעתי מהם קצת | ||||||||||
|
| |||||||||||
|
עלו כל הפרעונות כ"ח דינרי' וה' פשו' וע"ג חלקי' מצ"א בפשוט | ||||||||||
|
שיבא לכל פרעון ט' די' וה' פשוטי' ופ"ה חלקי' מצ"א | ||||||||||
|
ודרך עשיית החשבון הוא על זה הדרך שתתפוש החשבון אחר ותשים קצת הפרעון שבכל שנה ושנה ו' די' | ||||||||||
|
ותתפוש החשבון מסופו דהיינו שבסוף שנה שלישית נשארו ו' דינרי' | ||||||||||
|
ונרצה לדעת כמה נשארו בראש שנה שלישית שהוא סוף שנה שנייה | ||||||||||
|
אמור אם בסוף שנה שלישית נשארו ו' דינרי' הסר מהם השתות והנשאר הוא הקרן שנשאר בסוף שנה שנייה | ||||||||||
|
כי לעולם בכל חשבון עולה בו ריוח לחשבון דפה"ה החומש מלגין שהוא שתות מלבד | ||||||||||
|
ולכן כשתסיר ממנו [9]השתות ישאר הקרן לבד | ||||||||||
|
א"כ בראש שנה שלישית נשארו ה' די' | ||||||||||
|
ואז פרעת ו' די' עבור שנה שנייה הרי שבסוף שנה שנייה היו י"א | ||||||||||
|
הסר מהם השתות שהוא הריוח שעלה בהם בשנה שנייה נשארו ט' די' וב"פ | ||||||||||
|
ואז פרעת ו' די' בעבור שנה ראשונה הרי שבסוף שנה ראשונה היו ט"ו די' וב"פ | ||||||||||
|
הסר מהם השתות ישארו י"ב די' וז"פ וב' שלישי פשוט | ||||||||||
|
אם כן ידענו שאם הקרן הוא י"ב די' וז"פ וב' שלישי פשוט הפרעון הוא ו' די' בכל שנה ונרצה לידע אם הקרן הוא כ' די' כמה הוא הפרעון | ||||||||||
|
עשהו בדרך זה אם ג' שוה ד' ה' כמה שוה | ||||||||||
|
והבן זה כי עמוק הוא וכה"ל | ||||||||||
|
גם נוכל לעשותו בדרך אחרת | ||||||||||
|
ותאמר א"פ וב' שלשיות כמה חלק הוא מי"ב די' וז"פ וב' שלישיות הוא חלק אחד מצ"א | ||||||||||
|
אם תסירהו מן הקרן ישארו י"ב די' וחצי | ||||||||||
|
תסיר ממנו עוד מן הנשאר החומש ישארו י' די' | ||||||||||
|
כפלהו יעלה כ' די' | ||||||||||
|
וכן תעשה בפרעון תסיר מו' די' חלק אחד מצ"א אחרי כן תסיר מן הנשאר החומש ותכפול המותר | ||||||||||
| Pricing Problem - Find the Price | |||||||||||
|
מה פרי שקנית תגרות בכל כך ליט' הככר ותרצה לידע כמה יבא הליט' התגרות בדרך קצרה | ||||||||||
|
תפוש החומש מסכום החשבון המעות וד' חומשי' תשליך ומכל ליט' שנשאר בידך קח די' וכל כך מעות יבא הליט' מן התגרות | ||||||||||
|
המשל בזה הרי שקניתי ק' ליט' מפלפל לחשבון מ' ליט' ותרצה לידע כמה יבא הליט' | ||||||||||
|
תפוש החומש שהם ח' ליט' ומכל הליט' תקח די' הרי שיבא ח' די' הרי שיבא הליט' מן הפלפל ח' די' וה"ל | ||||||||||
|
אחר שבכל ליט' ממעות שהוציא בככר אחד מן התגרות יגיע לכל ליט' ב"פ וב' חמשי' | ||||||||||
|
והרי שקנית הככר מן התגרות במ' ליט' ותרצה לידע כמה יבא הליט' | ||||||||||
|
תאמר ב"פ מ"פ וב' פעמי' מ' חומשי פשוט הם ח' די' וכה"ל | ||||||||||
| Find the Earned Interest | |||||||||||
|
מו אם ישאלך אדם כמה יעלה הריוח מק' ליט' א' יום לחשבון ח' ליט' השנה | ||||||||||
|
תאמר ב' פעמי' ח' הם י"ו תפוש השליש בפרוטות שהם ה"פ ושליש וכן יבא וכה"ל | ||||||||||
Problems of Various Types |
|||||||||||
Rent Problem |
|||||||||||
|
מז אם תשכיר בית אחד לחשבון כך וכך ליט' השנה ותרצה לידע כמה יבא היום | ||||||||||
|
עשה כן מכל ליט' שתוציא בבית השנה יבא ליום ב' שלישי פשוט | ||||||||||
|
והרי שהשכרת בית אחד לחשבון כ' ליט' השנה | ||||||||||
|
תאמ' כ' פעמי' ב שלשיים הם מ' שהם י"ג פשו' ושלי' וכן יבא לזו | ||||||||||
Exchange Problems |
|||||||||||
|
מח אם ישאלך אדם הכ"ב פיסני שוים כ"ה בולייניני' כמה יתן חלוף הליט' מן הבולונייני' לליט' מן הפיסני' | ||||||||||
|
תרבה כ"ה פעמי' א' ליט' מבולנייני' יהיו כ"ה ליט' מבולנייני' חלקם על כ"ב יבא כ' די' וח"פ וח' חלקי' מי"א בפשוט | ||||||||||
Proportions of Fractions |
|||||||||||
|
מט אם שליש שוה רביע חומש כמה שוה | ||||||||||
|
אמור תחלה אנה ימצאו שליש ורביע וחומש אמור ג' פעמי' ד' י"ב | ||||||||||
|
השליש הוא כ' | ||||||||||
|
הרביע הוא ט"ו | ||||||||||
|
החומש הוא י"ב | ||||||||||
|
ואמור אם כ' שוה ט"ו י"ב כמה שוה | ||||||||||
|
אמור י"ב פעמי' ט"ו הם ק"פ חלקם בכ' חלקי' הרי שיבא ט' שהם ט' חלקי' מס' | ||||||||||
Find a Quantity Problem - Whole from Parts – Tree |
|||||||||||
|
[10]נ אם ישאלך אדם יש לי אי אילן שהחצי והשליש ממנו הוא עומד במים תחת הארץ והשליש והרבע מן הנשאר הוא מכוסה תקוע בארץ | ||||||||||
|
| |||||||||||
|
בקש המורה והוא ע"ב בכפלך ג' על ב' והם ו' וג' פעמי' ו' הם י"ח וד' פעמי' י"ח הם ע"ב | ||||||||||
|
ובע"ב תמצא חצי ושליש ושליש ורבע | ||||||||||
|
והוצא שליש וחצי מע"ב והם ס' וישארו י"ב | ||||||||||
|
א"כ בעבור החלק האחד שהוא בארץ הוצא השליש והרבע מי"ב והם ז' | ||||||||||
|
ועתה אסוף ז' עם ס' והם ס"ז וישארו ה' | ||||||||||
|
אם כן עשה אם ה' היו ג' ע"ב כמה היו | ||||||||||
|
ע"ב פעמי' ג' הם רי"ו | ||||||||||
|
חלקם בה' בא מ"ג וחומש | ||||||||||
|
א"כ אורך כל האילן היה מ"ג זרתות וחומש וכה"ל | ||||||||||
Motion Problem - To and From - an Ant Climbing a Tower |
|||||||||||
|
נא הרי שיש לפניך מגדל גבוה כ' אמה ונמלה אחת רוצה לעלות למעלה | ||||||||||
|
אמור תחלה כמה יותר השליש מן הרביע א' חלק מי"ב | ||||||||||
|
נמצא שבכל יום יש לה יתרון א' חלק מי"ב מן האמה | ||||||||||
|
הרי שבי"ב ימים היא עולה אמה אחת | ||||||||||
|
אמור י"ב פעמים כ' בעבור המגדל שגובהו כ' הרי ר"מ הרי שבר"מ ימים תעלה על המגדל | ||||||||||
Find a Quantity Problem - First from Last Problem – Money |
|||||||||||
|
נב הרי שיש לך מעות בכיס והוצאת מהם השליש והרביע והחומש והם ט' | ||||||||||
|
אמור תחלה אנה ימצאו שליש ורביע וחומש בס' | ||||||||||
|
השליש הוא כ' | ||||||||||
|
והרביע ט"ו | ||||||||||
|
והחומש י"ב | ||||||||||
|
וכללם מ"ז חלקי' מס' | ||||||||||
|
נמצא שהנשארי' הם י"ג חלקי' מס' | ||||||||||
|
ואמור אם מ"ז חלקי' מס' שוים ט' פשוט י"ג חלקי' מס' כמה יבואו | ||||||||||
|
אמור י"ג פעמי' ט' הם קי"ז חלקם במ"ז בא ב' וכ"ג חלקי' ממ"ז | ||||||||||
|
נמצא שהיו בתוך הכיס י"א וכ"ג חלקי' ממ"ז | ||||||||||
|
ומה שהוציא היו ט' ומה שנשאר היו ב' וכ"ג חלקי' ממ"ז וכה"ל | ||||||||||
|
נג אם ישאלך אדם היו לי מעות בכיס והוצאתי מהם העשירית ונשאר י' | ||||||||||
|
תפוש חשבון אחד ואמור מי' כשתקח ממנו העשירית ישארו ט' | ||||||||||
|
ואם כן הדרך היא כך אם ט' היו י' א' כמה היא | ||||||||||
|
כפול א' על י' הם י' חלקם על ט' יהיו א' ותשיעי' | ||||||||||
|
והוסיפם על הי' יהיו י"א ותשיעי' | ||||||||||
|
הוצא מהם העשירית ישארו י' שלמי' וכה"ל | ||||||||||
|
ואם יאמר כי נשארו י' ושתות | ||||||||||
|
עשה כן | ||||||||||
|
אחר שידעת כי האחר היא אחד ותשיעי' תאמ' אם אחת היא אחת ותשיעי' השתות כמה היא | ||||||||||
|
תפוש השתות מא' ותשיעי' | ||||||||||
|
והוסיפם על הי"א ותשיעי' וכן יצא | ||||||||||
Divide a Quantity – Money |
|||||||||||
|
נד אם תרצה לחלק ה"פ לשליש ולרביע ולא ישאר ממנו כלל | ||||||||||
|
אמור תחלה שליש ורביע נמצא בי"ב | ||||||||||
|
השליש הוא ד' | ||||||||||
|
הרביע הוא ג' | ||||||||||
|
חברם יחד הם ז' והוא המחלק | ||||||||||
|
ואם תרצה לידע כמה יבא למי שיש לו השליש שהוא ד' אמור ד' פעמי' ה' בעבור שהם הפ' יבא כ' | ||||||||||
|
ומי שהוא שיש לו הרביע שהוא ג' אמור ג' פעמי' ה' ט"ו | ||||||||||
|
חברם יחד יעלו ה' כמספר המעות הרי [11]שנחלקו לשליש ולרביע ולא נשאר מהם כלל וכה"ל | ||||||||||
Divide a Quantity – Money |
|||||||||||
|
נה אם תרצה לחלק י"ב פשוט לחצי ולשליש ולרביע | ||||||||||
|
אמור תחלה אנה ימצאו בי"ב | ||||||||||
|
החצי הוא ו' | ||||||||||
|
והשליש ד' | ||||||||||
|
והרביע ג' | ||||||||||
|
חברם הם י"ג והוא המחלק | ||||||||||
|
ומי שיש לו החצי אמור החצי מי"ב הוא ו' ואמור ו' פעמי' י"ב בעבור המעות שהם י"ב יבואו ע"ב | ||||||||||
|
ומי שיש לו השליש שהוא ד' אמור ד' י"ב הם מ"ח | ||||||||||
|
ומי שיש לו הרביע שהוא ג' אמור ג' פעמי' י"ב הם ל"ו | ||||||||||
|
ואם תאסוף כלם יבאו יב"פ כמספ' המעו' | ||||||||||
Ordering Problem - Six Coins |
|||||||||||
|
נו הרי שאדם יש לו שכיר אחד לשלשי' יום בל' פשו' ורוצה לקבל פרעונו בכל יום ואין לשוכר רק ו' מטבעות כסף ששוים ל"פ סך כולם | ||||||||||
|
סימ' א' ב' ג' ד' ח' י"ב | ||||||||||
Find a Number Problem - Ratio of Integers and Fractions |
|||||||||||
|
נז אם ג' ושליש שוים ד' ורביע ה' וחומש כמה שוים | ||||||||||
|
אמור ה' וחומש פעמי' ד' ורביע ומה שיבא חלקהו בג' ושליש | ||||||||||
|
וזה סדר עשייתו | ||||||||||
|
תפוש ה' וחומש ואמור ה' פעמי' כ"ה וא' הרי כ"ו הרי שעשית מה' וחומש כ"ו חמשיות ותזמן אותם כאשר תראה | ||||||||||
|
אחרי כן תפוש ד' ורביע ואמור ד' פעמי' ד' י"ו וא' הרי י"ז הרי שעשית מד' ורביע י"ז רבעיות | ||||||||||
|
ובעבור שאנו צריכים לומ' ה' וחומש פעמי' ד' ורביע תרבה כ"ו עם י"ז יעלו תמ"ב ונחלק אותם אחרי כן לרביע וחומש ונאמ' ד' פעמי' ה' כ' | ||||||||||
|
| |||||||||||
|
ובעבור שאנו צריכים לחלק בג' ושליש עשה מכ"ב וא' עשירי' כולם שלישייות ואמור ג' פעמי' כ"ב ס"ו וג' פעמי' א' עשירי' הם ג' עשריות הרי ס"ו וג' עשירייות | ||||||||||
|
חלק אותם אחרי כן בג' ושליש וזהו דרך חלוקו שתעשה מג' ושליש כולם שלישייות ואמור ג' פעמי' ג' ט' וא' הרי י' | ||||||||||
|
א"כ נחלק ס"ו בי' חלקי' יבואו ו' וו' עשירייות | ||||||||||
|
נחלק אחרי כן ג' עשיריות בי' חלקי' יבואו' ג' חלקי' מק' | ||||||||||
|
הרי שעולה החשבון ו' שלמים וו' עשירייות וג' חלקי' מק' | ||||||||||
|
נקבץ אותם יחד ונעשה מו' עשיריות חלקי' מק' ויהיו ס' וג' חלקי' שהיו לנו הרי ס"ג נמצא שיבא ו' שלמים וס"ג חלקי' ממאה | ||||||||||
|
| |||||||||||
Multiple Quantities - Boys Selling Eggs |
|||||||||||
|
נח אדם נתן לבניו ביצות למכור לאחד נתן נ' בצים ולשני ל' ולשלישי י' ואמ' להם לכו ומכרו הבצים בשוה וכולכם תביאו לי מעות בשוה | ||||||||||
|
הלכו ונתנו ז' בצים בפשוט | ||||||||||
|
אותו שהיו לו נ' בצים מכר מ"ט בצי' בז"פ ונשארה לו ביצה אחת | ||||||||||
|
ואותו שהיו לו ל' בצים מכר כ"ח בצים בד' פשו' ונשארו לו ב' בצים | ||||||||||
|
ואותו שהיו לו י' בצים מכר ז' בצים בפשו' ונשארו לו ג' בצים | ||||||||||
|
הילכו פעם שנייה ומכרו הבצים שנשארו להם בג"פ הביצה | ||||||||||
|
הראשון שנשארה לו ביצה אחת קבל ג' פשוט וז' שקבל במכירה ראשונה הרי י' פשו' | ||||||||||
|
והשני שנשארו לו ב' בצים קבל ו"פ וד"פ שקבל במכירה ראשונה הרי י"פ | ||||||||||
|
[12]והשלישי שנשארו לו ג' בצים קבל ט"פ וא"פ שקבל במכירה ראשונה הרי שקבל י"פ | ||||||||||
|
נמצא שכולם מכרו הבצים בשוה וכולם קיבלו מעות בשוה | ||||||||||
Find a Quantity Problem - Whole from Parts – Fish |
|||||||||||
|
נט הרי שיש לפניך דג ונחתך ראשו וזנבו והגוף שוקל י' ליט' | ||||||||||
|
אמור תחלה שליש ורביע וחומש ושתות נמצא בס' | ||||||||||
|
שהם כ' וט"ו וי"ב וי' שהם נ"ז חלקי' מס' | ||||||||||
|
נמצא שהראש והזנב ששקלו סך שניהם שליש ורביע וחומש ושתות הם נ"ז חלקי' מס' שהוא השלם | ||||||||||
|
| |||||||||||
|
ואותם הג' חלקי' שנשארו עד ס' הוא הגוף ששוקל י' ליט' | ||||||||||
|
אמור א"כ אם ג' חלקי' מס' שוקלים י' ליט' דהיינו הגוף הנשאר הנ"ז חלקי' מס' דהיינו השליש והרביע והחומש ושתות שהם הראש והזנב כמה שוקלי' | ||||||||||
|
אמור נ"ז פעמי' י' הם תק"ע | ||||||||||
|
חלקם בג' חלקי' יבוא ק"צ ליט' | ||||||||||
|
נמצא שהראש והזנב שוקלים ק"צ ליט' | ||||||||||
|
והגוף שוקל י' ליט' שהוא סך הכל ר' ליט' | ||||||||||
|
ואם תרצה לידע כמה שוקל הראש לבדו | ||||||||||
|
כבר ידעת כי הראש שוקל השליש והרביע והשליש והרביע מס' הם ל"ה חלקי' מס' | ||||||||||
|
ואמור אם ג' חלקי' מס' דהיינו הגוף שוקל י' ליט' אלו הל"ה חלקי' דהיינו הראש ששוקל שליש ורביע כמה שוקל | ||||||||||
|
אמור ל"ה פעמי' י' ש"נ | ||||||||||
|
חלקם בג' יבא קי"ו וב' שלשיות והוא משקל הראש | ||||||||||
|
ובזה הדרך תעשה גם הזנב ויצא לך הענין וכה"ל | ||||||||||
Simultaneous Division - Money in a Purse |
|||||||||||
|
ס ב אנשים היו להם מעות בא' כיס אמ' זה לזה שמעני אחי כך הם השליש והרביע ממעותי כמו השתות והשבע ממעותיך | ||||||||||
|
תמצא חשבון שימצא בו שליש ורביע וימצא בי"ב וכך מעות היו לו | ||||||||||
|
קח מהם שליש ורביע והם ז"פ | ||||||||||
|
ועבור האיש האחר שהיה לו שתות ושביע שהם י"ג חלקי' ממ"ב | ||||||||||
|
ואמור אם י"ג היו ז' כמה היו מ"ב | ||||||||||
|
אמור ז' פעמי' מ"ב רצ"ד חלקם בי"ג יהיו כ"ב וח' חלקי' מי"ג וכך מעות היו לו | ||||||||||
|
תוציא השתות והשביע מהם ויהיו ז"פ | ||||||||||
Partnership - for the same time |
|||||||||||
|
סא הרי שג' אנשים רצו להתחבר ולשים בחברותה מ' אונקי' זהב לאחד יש לו זהב ששוה ג' ליט' האונ' | ||||||||||
|
עשה על זה הדרך אמור שליש וחומש ושמינית אנה ימצאו בקש המורה והוא ק"כ | ||||||||||
|
השלשי הוא מ' | ||||||||||
|
החומש הוא כ"ד | ||||||||||
|
השמינית הוא ט"ו | ||||||||||
|
צרף כולם יהיו ע"ט והוא המחלק | ||||||||||
|
ואם תרצה לידע כמה זהב ישים אותו שיש לו הזהב ששוה ג' ליט' האונקי' אמור השלשי הוא מ' ותרבה אותו עם סך האונקי' שהם מ' ואמור מ' פעמי' מ' אלף ות"ר | ||||||||||
|
ואם תרצה לידע כמה שוה חלקו של זה אמור מן הכ' אונקי' יבוא ס' ליט' | ||||||||||
|
ומן הכ' חלקי' מע"ט אמור אם ע"ט שהוא אחד שלם שוה תש"כ פש' הכ' חלקי' מע"ט כמה שוים | ||||||||||
|
אמור כ' פעמי' תש"כ י"ד אלפי' ות' חלקם [13]בע"ט יבואו קפ"ב פשוטי' וכ"ב חלקי' מע"ט | ||||||||||
|
הרי ששוה חלקו של זה ס' ליט' וט"ו די' וב"פ וכ"ב חלקי' מע"ט | ||||||||||
|
וכן תעשה מן האחרים ויצא לך הענין וכה"ל | ||||||||||
Give and Take Problem - gatekeeper |
|||||||||||
|
סב איש אחד מהלך בד' עיירות ובעיר ראשונה הכפיל כל מעותיו ובצאתו מן העיר לקח לו השוער ח"פ | ||||||||||
|
אם תרצה לידע אותו עבור כי זה האיש הלך בד' עיירות ובכל עיר פרע ח"פ | ||||||||||
|
עבור העיר ראשונה צריך אלו הח"פ לחלק לחצי ויהיו ד"פ | ||||||||||
|
ועבו' העיר השנית אלו הד"פ חלק לחצי ויהיו ב"פ | ||||||||||
|
ועבו' העיר השלישית אלו הב"פ חלק לחצי ויהיה א"פ | ||||||||||
|
וזה הפשוט חלק לחצי ויהיה חצי פשוט | ||||||||||
|
ואסוף אחרי כן כולם יחד ויהיו ז' פשו' וחצי וכך היה הקרן וכה"ל | ||||||||||
|
|
|||||||||||
|
סג ד נהרות רצים אל מעיין אחד האחד ממלאו ביום אחד | ||||||||||
|
עשה על זה הדרך אמור ראשון וחצי ושליש ורביע ימצא בי"ב | ||||||||||
|
הראשון י"ב | ||||||||||
|
החצי ו' | ||||||||||
|
השליש ד' | ||||||||||
|
הרביע ג' | ||||||||||
|
חברם יחד הם כ"ה והוא המחלק | ||||||||||
|
הרי שבי"ב ימי' ימלאו כ"ה מעינות | ||||||||||
|
כיצד אותו שממלאהו ביום אחד א"כ בי"ב ימים ימלא י"ב מעינות | ||||||||||
|
ואותו שממלאהו בב' ימים א"כ בי"ב ימים ימלא ו' מעינות | ||||||||||
|
ואותו שממלאהו בג' ימים א"כ בי"ב ימים ימלא ד' מעיינות | ||||||||||
|
ואותו שממלאהו בד' ימים א"כ בי"ב ימים ימלא ג' מעיינו' | ||||||||||
|
נמצא שארבעתם יחד ימלאו בי"ב ימים כ"ה מעיינות | ||||||||||
|
אמור א"כ אם כ"ה מעיינות מתמלאי' בי"ב ימים א' מעיין בכמה מתמלא | ||||||||||
|
אמור א' פעם י"ב הם י"ב חלקם בכ"ה יבא י"ב חלקי' מכ"ה הרי שבי"ב חלקי' מכ"ה מתמלא ביום המעיין | ||||||||||
|
ואם תרצה לידע כמה חלק מן המים שם כל אחד מן הנהרות במעיין | ||||||||||
|
אמור הראשון הוא י"ב אמור י"ב פעמי' א' בעבו' המתמלא שהוא א' הם י"ב חלקם בכ"ה יבוא י"ב חלקי' מכ"ה | ||||||||||
|
ובזה הדרך תעשה מן האחרים וכה"ל | ||||||||||
|
ואם תרצה לידע כמה מעיינות ימלאו ביום אחד תאמ' א' פעם כ"ה הם כ"ה חלקם בי"ב יבואו ב' מעיינות וא' חלק מי"ב וכה"ל | ||||||||||
Motion Problem – Pursuit |
|||||||||||
|
סד הרי אדם אחד שהוא מהלך בכל יום י' מילין ואדם אחר מהלך ביום אחד א' מיל וביום שני ב' וביום ג' ג' והוא מוסיף בכל יום מיל אחד | ||||||||||
|
כפול י' עם י' ויבוא כ' תפחות א' מכ' יהיו י"ט הרי שבי"ט ימים יעמדו בשוה וכה"ל | ||||||||||
|
ואם הצולע מהלך אגה"ז ובכל יום מוסיף והולך פרדים ולא זוגות | ||||||||||
|
תפוס הי' מילים שמהלך הקבוע ודע שבי' ימים ישיגנו | ||||||||||
|
ואם הצולע מהלך בדו"ח וכן מוסיף והולך זוגות ולא פרדים | ||||||||||
|
תפחות א' מן הי' מילין שמהלך הקבוע [14]ואמור כי בט' ישיגנו ימים וכה"ל | ||||||||||
Mixture and Alligation Problem - Goldsmith making rings from several kinds of coins |
|||||||||||
|
סה הרי שנתתה לצורף י' אונקיו' זהב לעשות ממנו טבעות והב' אונקיו' מהם היה זהב מי"ד קראטי' האונקי' | ||||||||||
|
תפוש סכום האונק' והקרטי' וכפול הקרטי' בס | ||||||||||
|
כיצד הב' אונ' מי"ד קרטי' האונ' ת..ם כ"ח | ||||||||||
|
והג' אונק' מי"ו קרטי' האונ' ת..ם מ"ח | ||||||||||
|
והה' אונק' מי"ח קרטי' האונ' ת..ם צ' | ||||||||||
|
חברם יחד הם קס"ו קרטי' | ||||||||||
|
חלקם לי' חלקי' כמנין האונ' יבא לכל אונק' י"ו קרטי' וג' חומשי קרטי' וכה"ל | ||||||||||
Divide a Quantity - Price of a Fish Paid by a Group of People |
|||||||||||
|
סו הרי שג' אנשים קנו א' דג בט"פ לאחד יש לו החצי ולשני יש לו השליש ולשלישי יש לו התשיעי | ||||||||||
|
עשה על זה הדרך אמור אנה ימצא חצי ושליש ותשיעי בי"ח | ||||||||||
|
החצי הוא ט' | ||||||||||
|
השליש הוא ו' | ||||||||||
|
התשיעי הוא ב' | ||||||||||
|
חברם יחד הם י"ז והוא המחלק | ||||||||||
|
ואותו שיש לו החצי מן הדג אמור החצי הוא ט' | ||||||||||
|
ותרבה אותו על הדג שהוא א' ואמור ט' פעמי' א' הוא ט' חלקהו בי"ז יבא לחלקו ט' חלקי' מי"ז | ||||||||||
|
וכן תעשה מן האחרים ויצא לך הענין וכה"ל | ||||||||||
Joint Purchase Problem - If You Give Me - two men - Amounts of Money |
|||||||||||
|
סז אם יאמר אדם לחבירו תן לי ו"פ יהיה לי כאשר יש לך והאחר אמ' אם תתן לי ו"פ יהיה לי פי שנים ממך | ||||||||||
|
תאמ' ו' פעמי' ז' מ"ב וכך מעות היו לאיש שאמר תן לי ו"פ יהיה לי כפול ממך | ||||||||||
|
ולמעות האחר תאמ' ה' פעמ' ו' ל' וכן היו לו כה"ל | ||||||||||
Interest and Discount Problem - find the fund |
|||||||||||
|
סח אם נאמר לך אני פלני עשיתי מא"פ ב' ומב' עשיתי ג' ומג' ד' ומד' ה' ומה' ו' והיו לי בין קרן וריוח ת"פ אשאלך כמה היה הקרן | ||||||||||
|
עשה כן עת' כי מאחד עשית ב' ומב' ג' ומג' ד' ומד' ה' ומה' ו' א"כ צריך לראות כי ו' יצאו מא' א"כ מאיין מצים לצאת ת"פ | ||||||||||
|
עשה כן א' פעם ת"פ וחלקם בו' יהיו ס"ו פשו' וב' שלישי פשוט וכן היה הקרן | ||||||||||
Exchange Problems |
|||||||||||
|
סט כ די' אונקוניטני לחילוף בולייניני' לא"פ וחצי הליט' שוה פחות מן הבולונייני' כמה יבא לל"ז די' וחצי מאנקונטני' מחלוף | ||||||||||
|
עשה כן ל"ז פעמי' ל"א הם אלף וקמ"ז | ||||||||||
|
ואחר אמור איזהו החצי מל"א הוא ט"ו וחצי | ||||||||||
|
ומהו החצי מל"ז והוא י"ח וחצי | ||||||||||
|
ומהו החצי מן החצי הוא רביע | ||||||||||
|
ואסוף ט"ו וחצי וי"ח וחצי ורביע יהיו אלף וקפ"א ורביע חלק אותם לכ' חלקי' יבא נ"ט פשו' וא' חלק מכ' וא' חלק מפ' | ||||||||||
|
| |||||||||||
|
ואם יאמר לצ"א די' ורביע כמה יבא כמו כן | ||||||||||
|
תרבה צ"א פעמי' ל"א והם אלפיים ותתכ"א | ||||||||||
|
ואחר אמור איזהו הרביע מל"א הוא ז' וג' רבעי' | ||||||||||
|
ומה הוא החצי מצ"א והוא מ"ה וחצי | ||||||||||
|
ומהו החצי מן הרביע הוא שמינית | ||||||||||
|
ואסוף עם אלפים ותתכ"א ויהיו אלפי' ותתע"ד וא' רביע וא' שמינית וחלקם בכ' חלקי' ויהיו [15]קמ"ג פשו' וג' רבעים וא' חלק מכ' | ||||||||||
|
| |||||||||||
Interest and Discount Problem |
|||||||||||
|
ע חשבון אחר שבין קרן וריוח היו בשנה אחת י"ז ליט' לחשבון גפה"ה כמה היה הקרן | ||||||||||
|
תפוס חשבון אחד ואמור כ"ג ליט' לחשבון גפה"ה היו בסוף השנה כ' ליט' מקרן וג' ליט' מריוח | ||||||||||
|
ואמור אם כ"ג היה כ' י"ז כמה היה | ||||||||||
|
אמור י"ז פעמי' כ' ש"מ חלקם על כ"ג יהיו י"ד ליט' וט"ו די' נוס' וט"ו חלקי' מכ"ג בפשוט וכה"ל | ||||||||||
Pricing Problem - Find the Amount |
|||||||||||
|
עא אם נשאלת בכ"א ליט' וחצי הככר מן העורות עבור י"ב ליט' ורביע כמה עורות יבא | ||||||||||
|
עשה כ"א וחצי וכ"א וחצי ויהיו מ"ג וזהו המחלק | ||||||||||
|
ואחר עשה ב' פעמי' ק' והם מאתיי' | ||||||||||
|
ותרבה י"ב ליט' ורביע עם מאתיים ויהיו אלפיי' ות"נ וחלק במ"ג חלקים ויהיו נ"ו עורות ומ"ב חלקי' ממ"ג מא' עור וכה"ל | ||||||||||
|
ואם יאמר בע"א ליט' וחצי כמה עורות יבואו לו כמו כן | ||||||||||
|
תרבה ע"א ליט' וחצי עם ר' ויהיו קמ"ג ככרים וחלק במ"ג ויהיו של"ב עורות וכ"ד חלקי' ממ"ג מא' עור וכה"ל | ||||||||||
Divide a Quantity - Sharing Food |
|||||||||||
|
עב ב אנשים היו יושבים לאכול לאחד יש לו ב' לחמים ולשני ג' | ||||||||||
|
אמור כמה חלקי' מן הלחם אכל כל אחד ואחד א' לחם וב' שלישיות | ||||||||||
|
א"כ אותו שהיו לו ב' לחמים ואכל א' לחם וב' שלשיות לא הפסיד כי אם שליש לחם | ||||||||||
|
ואותו שהיו לו ג' לחמים ואכל א' לחם וב' שלשיות הפסיד א' לחם ושליש דהיינו ד' שלשיות | ||||||||||
|
א"כ אותו שהיו לו ב' לחמי' יקבל א"פ | ||||||||||
|
והאחר יקבל ד"פ | ||||||||||
Find a Number Problems - Multiplication of fractions |
|||||||||||
Fractions by Fractions |
|||||||||||
|
עג אם ישאלך אדם כמה הוא ד' חמשיות על ד' חמשיות | ||||||||||
|
תאמר אם היה שואל א' חמישית על א' חמישית | ||||||||||
|
הייתה אומר ה' פעמי' ה' כ"ה והיה עולה א' חלק מכ"ה | ||||||||||
|
ועתה שהוא שואל ד' חמשיות על ד' חמשיות עדיין תאמ' ד' פעמי' ד' י"ו נמצא שיבא י"ו חלקי' מכ"ה | ||||||||||
|
עד אי"א כמה הוא ז' תשיעיות על ד' שבעיות | ||||||||||
|
תאמ' אם היה שואל א' תשיעית על א' שבעית | ||||||||||
|
הייתה אומ' ז' פעמ' ט' הם ס"ג נמצא שהיה עולה א' חלק מס"ג | ||||||||||
|
ועתה שהוא שואל ז' תשעיות על ד' שבעיות עדיין תאמ' ז' פעמ' ד' כ"ח נמצא שיבא כ"ח חלקי' מס"ג וכה"ל | ||||||||||
Integers and Fractions |
|||||||||||
|
אי"א ג' שלמים וב' חמשיות על ב' שלמים וד' שבעיות כמה הוא | ||||||||||
|
תאמ' ג' פעמ' ב' הם ו' שלמים | ||||||||||
|
אח"כ תאמר ג' פעמ' ד' שבעיות הם י"ב שהוא אחד שלם וה' שבעיות שהוא כ"ה חלקי' מל"ה | ||||||||||
|
אח"כ תאמ' ב' פעמ' ב' חמשיות הם ד' חמשיות שהם כ"ח חלקי' מל"ה | ||||||||||
|
ואחרי כן תאמ' ב' חמשיות על ד' שבעיות הוא ח' חלקי' מל"ה | ||||||||||
|
צרף כלם יחד יבא ח' שלמים וכ"ו חלקי' מל"ה וכן יבא וכה"ל | ||||||||||
|
| |||||||||||
|
עה אי"א א' וה' שבעיות על א' וד' חמשיות כמה הוא | ||||||||||
|
אמור א' פעם א' א' | ||||||||||
|
א"כ תאמ' [16]א' פעם ד' חמשיות הם כ"ח חלקי' מל"ה | ||||||||||
|
אח"כ תאמ' א' פעם ה' שבעיות הם כ"ה חלקי' מל"ה | ||||||||||
|
אחר כן תאמ' ד' חמשיות על ה' שבעיות הם כ' חלקי' מל"ה | ||||||||||
|
צרפם יחד יבא ג' שלמים וג' חלקי' מל"ה וכה"ל | ||||||||||
|
| |||||||||||
Multiple Quantities - Coins that Worth an Amount of Money |
|||||||||||
|
עו הרי שיש לך ד' מטבעות ששוים סך ארבעתם פ' פשוטי' והראשון שוה החצי והשתות מן השני | ||||||||||
|
| |||||||||||
|
עשה על זה הדרך | ||||||||||
|
אמור הראשון ששוה החצי והשתות מן השני | ||||||||||
|
אמור חצי ושתות אנה ימצא בו' | ||||||||||
|
החצי הוא ג' השתות הוא א' הרי ד' חלקי' מו' שהם ב' שלשיות | ||||||||||
|
| |||||||||||
|
א"כ זה ששוה חצי ושתות מן השני הוא כאלו אמר הראשון שוה ב' שלשיות מהשני | ||||||||||
|
והשלשי ששוה שליש ורביע ושתות מן הראשון | ||||||||||
|
אמור אנה ימצא שליש ורביע ושתות בס' | ||||||||||
|
השליש הוא כ' הרביע ט"ו והשתות י' הרי למ"ה | ||||||||||
|
| |||||||||||
|
א"כ השלשי ששוה שליש ורביע ושתות מן הראשון שהם מ"ה חלקי' מס' הוא כאלו אמר ג' רבעיות מן הראשון | ||||||||||
|
והרבעי ששוה ד' חמשיות מהשלשי הוא מבואר | ||||||||||
|
א"כ הסדר כך הוא הראשון ששוה ב' שלשיות מהשני והשלשי שוה ג' רבעיות מן הראשון | ||||||||||
|
| |||||||||||
|
אמור תחלה השברים הנז' בזה החשבון הם חצי ושליש ורבע וחומש ושתות וימצאו כלם בס' | ||||||||||
|
א"כ השני הוא ס' | ||||||||||
|
והראשון שהוא שוה ב' שלשיות מן השני שהוא ס' הוא מ' כי מ' הוא ב' שלשיות מס' | ||||||||||
|
והשלשי ששוה ג' רבעיות מהראשון שהוא מ' א"כ הוא ל' כי ל' הם ג' רבעיות ממ' | ||||||||||
|
והרבעי ששוה ד' חמשיות מהשלשי שהוא ל' הוא כ"ד כי כ"ד הוא ד' חמשיות מל' | ||||||||||
|
| |||||||||||
|
והנשאר מהשני שהוא ב' שלשיות מהשלשי עשה על זה הדרך | ||||||||||
|
אמור כמה נשאר מן השני שהוא ס' אחר שהוצאת ממנו הראשון שהוא מ' נשארו כ' | ||||||||||
|
א"כ כ' שהוא הנשאר מהשני הם ב' שלשיות מן השלשי שהוא ל' כי כ' הם ב' שלשיות מל' | ||||||||||
|
הרי שהראשון הוא מ' והשני הוא ס' והשלשי ל' והרבעי כ"ד צרף אותם יחד יעלו קנ"ד והוא המחלק | ||||||||||
|
ואם תרצה לידע כמה שוה הראשון שהוא מ' אמור מ' פעמ' פ' בעבור שכלם שוים פ' יהיו ג' אלפי' ומאתיים | ||||||||||
|
ובזה הדרך תעשה מן האחרים ויצא לך הענין השוה וכה"ל | ||||||||||
Find a Number Problems - Sums |
|||||||||||
|
עז א ב' ג' ד' ה' ו' ז' ח' | ||||||||||
|
שהם נחלקי' לחצי | ||||||||||
|
תוסיף א' על הסך ותרבה אותו על החצי | ||||||||||
|
ואמור ד' פעמ' ט' ל"ו וכן יהיה מספרם ל"ו וכה"ל | ||||||||||
|
עח א ב' ג' ד' ה' ו' ז' | ||||||||||
|
שאינם נחלקים לחצי | ||||||||||
|
תפוס הרוב ותרבה אותו על הסך | ||||||||||
|
ואמור ד' פעמ' ז' כ"ח וכה"ל | ||||||||||
|
עט א ג' ה' ז' ט' שהם פרדים | ||||||||||
|
תרבה הרוב בעצמו | ||||||||||
|
ואמור הרוב מט' הוא ה' ואמ' ה' פעמ' ה' כ"ה וכן יבאו וכה"ל | ||||||||||
|
פ ב ד' ו' ח' שהם כלם זוגות | ||||||||||
|
תרבה חציים על הבא לאחריו | ||||||||||
|
ואמור החצי מח' ד' [17]ואמור ד' פעמ' ה' הם כ' וכן יבא וכה"ל | ||||||||||
Find a Number Problem - Multiplication of integers and fractions |
|||||||||||
|
פא אם ישאלך אדם א' ורביע על א' וחמישית כמה הוא | ||||||||||
|
אמור תחלה א' פעם א' א' | ||||||||||
|
אח"כ תאמ' פעם אחת רביע ופעם אחת חמישית הם ט' בנטיני' | ||||||||||
|
אחר כך תאמ' א' רביע על א' חמישית הוא א' חלק מכ' | ||||||||||
|
נמצא שיבא הכל א' וי' בנטיני' דהיינו א' וחצי | ||||||||||
Interest and Discount Problems |
|||||||||||
Find the Earned Interest |
|||||||||||
|
פב אם ישאלך אדם הלותי מעות לחשבון כך וכך פשו' החדש הליט' כמה יעלה ריוח בק' ליט' א' שנה | ||||||||||
|
תרבה ה' פעמ' כנגד המעות שירויח הליט' החדש | ||||||||||
|
המשל בזה הרי שהלוית ק' ליט' א' חדש לחשבון ז"פ החדש הליט' | ||||||||||
|
תאמ' ה' פעמ' ז' ל"ה | ||||||||||
|
נמצא שיבא הק' ליט' השנה ל"ה ליט' וכה"ל | ||||||||||
Compound Interest |
|||||||||||
|
פג) מי שרוצה לקבץ חשבונות הלוואות רבים שנעשו בזמנים שונים להביאם בזמן אחד | ||||||||||
|
יקבץ חשבונות החדשים כלם ויחלקם כחשבון המעות או הפרחי' או כמטבע ההלוואות ההם | ||||||||||
|
המשל בזה הרי שהלוית ק' פרחי' זהב א' חדש וק' פרחי' ב' חדשים וק' פרחי' ג' חדשי' וק' פרחי' ד' חדשי' וק' פרחי' ה' חדשי' | ||||||||||
|
עשה כן אחד ושנים ושלשה וארבעה וחמשה שהם החדשי' הרי ט"ו | ||||||||||
|
חלקם בסכום המעות שהם ה' יגיע לכל חלק מהם ג' | ||||||||||
|
הרי שעמדו כלם ג' חדשי' | ||||||||||
|
וכן הדומה לכל מטבע ולכל מנין וכה"ל | ||||||||||
Find the Earned Interest |
|||||||||||
|
פד הרוצה לידע לחשבון כך וכך הככר כמה יבואו קצתם לחדש | ||||||||||
|
תרבה חשבון הככר כסכום המעות שתרצה וחלקם בחמשה והחלק שיצא יגיע לכל חדש כסכום ההוא | ||||||||||
|
המשל בזה לחשבון ז' ליט' הככר לשנה כמה יבא ל' ליט' חדש אחד או ב' חדשי' או יותר | ||||||||||
|
תרבה ל' בז' והם ר"י | ||||||||||
|
חלקם בחמשה יגיע לכל חלק מהם מ"ב | ||||||||||
|
הרי שבמב"פ יבא לכל לחדש אחד | ||||||||||
|
אחרי כן תרבה החדשים שתרצה ויצא לך הענין וכה"ל | ||||||||||
Multiple Quantities Problem - Five Weights |
|||||||||||
|
פה אם הקצב מוכר בשר ואין לו רק ה' משקלות ששוקלות בין כולם קכ"א ליט' כמה ישקול כל משקל לעצמו שיוכל הקצב לשקול הבשר באלו המשקלות למי רב ולמי מעט | ||||||||||
|
שמי שירצה לקנות קכ"א ליט' בשר ישים כל המשקלות כנגד הבשר | ||||||||||
|
ואם ירצה לקנות נ' ליט' בשר או יותר או פחות ישים כל כך משקלות בצד המאזנים שכנגד הבשר שישקלו כמו הבשר שהוא רוצה לקנות | ||||||||||
|
ואם לא יבא בכיוון יחליף המשקלות | ||||||||||
|
ולפעמים צריך שישים אחד ושנים ושלשה משקלות עם הבשר כנגד משקל אחד להפיק רצון הקונה | ||||||||||
|
ועתה אשאלך כמה ישקול כל משקל לעצמו | ||||||||||
|
האחד ישקול א' ליט' | ||||||||||
|
והשני ישקול ג' ליט' | ||||||||||
|
והשלישי ט' ליט' | ||||||||||
|
והרבעי כ"ז ליט' | ||||||||||
|
והחמשי פ"א ליט' | ||||||||||
|
צרפם יחד יהיו קכ"א ליט' לא פחות ולא יותר | ||||||||||
|
ובאלו המשקלות יוכל הקצב למכור כרצונו למי רב ולמי מעט וכה"ל | ||||||||||
Guessing - coins |
|||||||||||
|
פו חשבון הג' מטבעות שונות לג' אנשים שונים להגידם לראשון תן א"פ ולשני ב' ולשלשי ד' ובעל המטבע הגדול יכפול חלקו ד' פעמ' והשני ג' פעמים [18]ובעל המטבע הקטן יכפול חלקו פעם אחד | ||||||||||
|
וסימן א' כסף זהב נחשת | ||||||||||
|
ב' זהב נחשת כסף | ||||||||||
|
ג' זהב כסף נחשת | ||||||||||
|
ד' נחשת כסף זהב | ||||||||||
|
ה' נחשת זהב כסף | ||||||||||
|
ו' כסף נחשת זהב וכה"ל | ||||||||||
Payment Problem - three workers, three different daily wages, the same actual payment |
|||||||||||
|
פז אדם שכר ג' אחים ראובן שמעון לוי שיעשו עבודתו כ' ימים מן הבקר עד הערב מאיזה מהם שיהיה ולא תשבות המלאכה והנה אם עבד ראובן כל הימים יתן לו ה' זהובים ואם שמעון ד' ואם לוי ג' | ||||||||||
|
דע כי ראובן ישמש ד' ימים בזהוב אחד | ||||||||||
|
ושמעון ה' ימים | ||||||||||
|
ולוי ו' ימים וב' שלישיות יום | ||||||||||
|
והנה הכל ט"ו ימים וב' שלישיות אחד | ||||||||||
|
נחלק כ' על זה המספר ועלה אחד שלם ונשארו ד' שלמים ושלישית | ||||||||||
|
והנה בעבור השלישית נשים הכל שלישיות | ||||||||||
|
והנה נשיב הכ' ימים ס' שלשיות והט"ו וב' שלישיו' מ"ז שלשיות והד' ושלישית י"ג | ||||||||||
|
והנה כל אחד לקח זהוב אחד וי"ג פשו' ממטבע מ"ז בזהוב | ||||||||||
|
| |||||||||||
|
ועתה נבקש כמה חייב כל אחד לעבוד עד שישלימו הכ' ימים | ||||||||||
|
והנה נחל מלוי שחייב לשמש בזהוב שלקח ו' ימים וב' שלישיות | ||||||||||
|
ונעשה מאלו שלשיות ויהיו כ' | ||||||||||
|
ונבקש לדעת כמה יש לו לעבוד בעבור יג"פ שלקח | ||||||||||
|
כפלנו י"ג על כ' היו ר"ס | ||||||||||
|
חלקנום על מ"ז עלו ה' נשארו כ"ה חלקי' | ||||||||||
|
חברנו ה' עם כ' היו כ"ה שלשיות וכ"ה חלקי' ממ"ז | ||||||||||
|
חלקנו אלו השלישיות על ג' עלו ח' שלמים ונשאר אחד | ||||||||||
|
נקח לו ד' שעות שהם שלישית יום | ||||||||||
|
נכפול כ"ה על ד' עלו ק' | ||||||||||
|
נחלקם על מ"ז עלו ב' שעות ונשארו ו' חלקי' | ||||||||||
|
| |||||||||||
|
והנה לוי עבד ח' ימים ו' שעות ו' חלקי' | ||||||||||
|
נבקש לדעת כמה עבד שמעון הוא חייב לעבוד בעבור הזהוב ה' ימים שהם ט"ו שלישיות | ||||||||||
|
ונבקש לדעת כמה יעבוד בעבור יג"פ שלקח | ||||||||||
|
נכפול י"ג על ט"ו עלו קצ"ה | ||||||||||
|
נחלקם על מ"ז יעלו ד' ונשאר ז' חלקי' | ||||||||||
|
נחבר הד' אל הט"ו כי שלשיות הם יהיו י"ט שלשיות | ||||||||||
|
נחלקם על ג' יהיו ו' ימים שלמים | ||||||||||
|
ונקח לאחד הנשאר ד' שעו' | ||||||||||
|
גם נכפול ז' על ד' יהיו כ"ח | ||||||||||
|
| |||||||||||
|
והנה הם ו' ימים וד' שעות וכ"ח חלקי' וככה עבד שמעון | ||||||||||
|
נבקש לדעת כמה עבד ראובן והנה עבד בשביל הזהוב ד' ימים שהם י"ב שלשיות | ||||||||||
|
ונעשה בעבור היג"פ שלקח הערך | ||||||||||
|
|||||||||||
|
נחלקם על מ"ז עלו ג' ונשארו ט"ו חלקים | ||||||||||
|
חברנו אלו הג' עם הי"ב שהיו לנו היו ט"ו והם שלשיות | ||||||||||
|
והנה עבד ה' ימים | ||||||||||
|
גם הט"ו חלקי' נכפול על ד' עלו ס' | ||||||||||
|
נחלקם על מ"ז עלתה שעה אחת ונשארו י"ג חלקי' משעה שעבד ראובן | ||||||||||
|
| |||||||||||
|
וכאשר תחבר אלה החלקי' יעלה מהם שעה אחת בלי תוספת ומגרעת | ||||||||||
|
וכאשר תחבר שעה זו לשעות הנזכרות יהיו י"ב שעות שהוא יום אחד | ||||||||||
|
וכאשר תחבר היום לימים הנז' יהיו כ' ימים בלי תוספת ומגרעת וכה"ל | ||||||||||
|
| |||||||||||
Divide a Quantity Problem - Proportional Division – Inheritance |
|||||||||||
|
פח שאלה יעקב מת והוציא ראובן שטר בשני עדים כשרים שנתן לו יעקב אביו כל [19]הממון שהיה לו וציוה כן מחמת מיתה | ||||||||||
| Three methods to divide the property between the four sons each according to his relative portion: | |||||||||||
|
והנה חכמי ישראל מחלקים אותו על דרך בקשת כל אחד | ||||||||||
|
וחכמי הגוים על דרך ערך הממון של כל אחד | ||||||||||
|
וחכמי החשבון יחשבו כי הממון היה אחד וכאשר תחבר אליו חציתו ושלשיתו ורבעיתו יהיו הכל שנים וחצי ששית | ||||||||||
|
והנה נשים האחד שלם ס' שיש לו כל החלקי' הנז' ויהיו בין הכל קכ"ה | ||||||||||
|
או נשים האחד שלם י"ב והשברים הנז' י"ג | ||||||||||
|
ושוה יצא המספר באחרונה אי זה מהם שתקח | ||||||||||
|
ועתה נבקש כמה יקח ראובן לפי ערך ממונו ונעשה הערך ככה על דרך ס' כי הוא מבקש כל הממון ונעשה כי הממון י' די' שהם ק"כ פ' | ||||||||||
|
וזה צורת ערך הממון שיקח ראובן | ||||||||||
|
| ||||||||||
|
כפלנו הקצוות עלו ז' אלפי' ור' חלקנום על קכ"ה עלו נ"ז פשו' וע"ה חלקי' וזה חלק ראובן | ||||||||||
|
וזה צורת ערך שמעון | ||||||||||
|
| ||||||||||
|
נכפול הקצוות ונחלק כמשפט | ||||||||||
|
וזה צורת חלק לוי | ||||||||||
|
| ||||||||||
|
ונכפול ונחלק כמשפט | ||||||||||
|
וזו צורת חלק יהודה | ||||||||||
|
| ||||||||||
|
ונכפול ונחלק כמשפט | ||||||||||
|
ענין אחר בדרך קצרה | ||||||||||
|
יקח שמעון חצי חלק ראובן | ||||||||||
|
ולוי יקח שליש חלק ראובן | ||||||||||
|
ויהודה יקח רביע חלק ראובן | ||||||||||
|
וכאשר תחבר אלו כל החלקי' והשלמים יהיו ק"כ פ' שהם י' די' | ||||||||||
|
ועל דרך חכמי ישראל | ||||||||||
|
יאמר הג' אחים הגדולים ליהודה אין אתה מערער רק על ל"פ וערעור כל אחד ממנו שוה בהם קח ז' וחצי שהוא הרבעית ולך מעמנו | ||||||||||
|
וכמו כן יקח כל אחד מהג' אחים | ||||||||||
|
ועוד יאמר ראובן ללוי אין אתה מערער רק על מ"פ וכבר לקחת חלקך מהל' שהארבעתינו חלקנו עליו קח אתה שלישית י' שהוא רבעית מ' ולך מעמנו | ||||||||||
|
והנה חלק לוי י' וה' ששיות פשוטי כי החצי מן הז' וחצי שלקח כבר הם ג' ששיות ושליש אחד מן הי' הם ב' ששיות הרי ה' ששיות | ||||||||||
|
| |||||||||||
|
גם ראובן יאמ' לשמעון אין אתה מערער רק על חצי האחד שהוא ס' וחצי האחר כולו שלי וכבר לקחת חלק מהמ' והנה שנשאר בינך ובני הערעור קח חציים ולך מעלי | ||||||||||
|
והנה חלק שמעון כ' וה' ששיות פשוט | ||||||||||
|
| |||||||||||
|
וחלק ראובן פ' וה' ששיות פשוט | ||||||||||
|
| |||||||||||
|
וכאשר תחבר אלו החלקי' יהיו י' די' וכה"ל | ||||||||||
Geometrical Problems |
||
Triangulation Problem - Two towers |
||
|
פט ב מגדלים אחד גבוה ט"ו אמות ואחד גבוה י"ב אמות ושם ב' יונים ומעיין אחד ביניהם ומגיעים ברגע אחד במעיין | |
|
|
||
|
שים הרוחק שבניהם כמדת גובה שתיהם דהיינו י"ב וט"ו שהם כ"ז | |
|
וחלק כ"ז לי"ב ולט"ו | |
|
ושים הט"ו אצל המגדל שהוא גבוה י"ב | |
|
ושים הי"ב אצל המגדל שהוא גבוה ט"ו | |
|
ובגבול הט"ו והי"ב מן התושבת שם המעיין וכה"ל | |
Rectangle |
||
| Figure Problem - Side; Diagonal; Area – Rectangle | ||
|---|---|---|
|
צ שאלה מרובע ארוך אשר אלכסונו עם צלעו האחד י"ח וצלעו השנית ו' כמה הוא ריבועו ואלכסונו וצלעו המנויה עם האלכסון | |
|
||
|
והמשיב על השאלה הזאת [20]ירבע את הצלע הידועה והוא ו' ויהיה רביעו ל"ו | |
|
ויחלקם על מניין האלכסון והצלע השנית שהוא י"ח ותהיה החלוקה ב' | |
|
ויוסיף ב' על י"ח ויהיו כ' | |
|
וידע כי מחצית כ' הוא האלכסון | |
|
| ||
|
והנשאר מי"ח הוא הצלע השנית והוא ח' | |
|
ורבועם ח' בו' והוא התשבורת | |
Quadrangle |
||
| Figure Problem - Area - Quadrangle | ||
|
צא הרי שיש לפניך א' מרובע שאין ארכו כרחבו ואינו שוה האורך האחד לאורך השני וגם רוחב האחד אינו שוה לרוחב השני | |
|
צרף האורך וחלקהו לחצי ותפוש חציו | |
|
וצרף הרוחב עם הרוחב ותפוש חציו | |
|
ותרבה החצי משני הארכים על החצי משני הרחבים וכפי מספר אותם הרבויים יהיו מספר שבריו | |
|
| ||
|
||
|
והמשל בזה הרי שהיה מקום אחד ארכו האחד ד' ואורכו השני ו' ורחבו האחד ה' ורחבו השני ג' כזה | |
|
|
||
|
צרף האורך עם האורך ואמ' ו' וד' הרי י' תפוס חציו הרי ה' ויהיה ד' ארכו ו' לך למשמר | |
|
אחרי כן צרף הרוחב עם הרוחב ואמור ג' וה' הרי ח' תפוס חציו שהוא ד' רחבו ה' | |
|
ותרבה אותו עם הה' שהוא מחצית ב' האורכים ואמור ד' פעמ' ה' הם כ' הרי שמידת שבריו הם כ' וכה"ל | |
|
| ||
Triangulation Problem - Tree leaning against a wall |
||
|
צב הרי שעץ אחד גבוה ל' אמות וזקוף בצד חומה אחת והוסר מלמטה י' אמות נרצה לידע מזה כמה הורד ראש העץ ממקום שהיה | |
|
||
|
אתה יודע כי החומה דהיינו מראש העץ עד הקרקע והקרקע דהיינו מן החומה עד סוף העץ הם שני זוויות על זוית נצבה | |
|
||
|
והאלכסון שלהם הוא העץ שהוא ל' אמות ושברי מרובע שיוצא ממנו הם תת"ק | |
|
ואחר כן תרבע קו הקרקע שהוא י' ושבריו הם ק' | |
|
והוציאם מן התת"ק ישארו ת"ת | |
|
ומצא השורש והוא קו החומה ויהיה מעט יותר מכ"ח ורביע | |
|
| ||
|
והוציאם מן הל' שהיה קו החומה כשהיה העץ זקוף ישארו מעט פחות מא' וג' רבעי' וכן הורד העץ | |
|
ולפעמי' יבא בכיוון וכה"ל | |
|
צג וכן אם היה עץ אחד גבוה י' אמות וזקוף בצד חומה והוסר מלמטה ו' אמות כמה הורד ראש העץ ממקום שהיה | |
|
||
|
עשה כמו שעשינו למעלה ותרבע מדת העץ והם ק' | |
|
אחר כן תרבע הו' אמות שהורד מלמטה והם ל"ו | |
|
והוציאם מן הק' ישארו ס"ד | |
|
ומצא השורש דהיינו ח' והוא קו החומה | |
|
| ||
|
והוציאם מן הי' שהיה קו החומה כשהיה העץ זקוף ומה שישאר בידך הורד העץ דהיינו ב' אמות | |
|
ועתה בא בכיוון וכה"ל | |
Transformation Problems |
||
| Square to Circle | ||
|
צד מרובע שוה צלעות ושבריו ל"ח וחצי ותרצה לעשות מהם עגול שיהיו שבריו כשברי זה המרובע | |
|
| ||
|
תרבה ל"ח וחצי על י"ב וד' שבעיות עולים תפ"ד | |
|
ותמצא השורש מתפ"ד והם כ"ב והוא יהיה מספר העגול יהיו שבריו כשבר המרובע שמספר שבריו ל"ח וחצי וכה"ל | |
|
| ||
| Circle to Square | ||
|
צה עגול שבריו ק' ותרצה לעשות ממנו מרובע שוה צלעות | |
|
| ||
|
תפוס השורש מק' והוא י' והוא יהיה מדת כל צלע מצלעו וכה"ל | |
Volume |
||
Sphere |
||
|
[21]צו תשבורת הכדור | |
|
כאשר זכרו אנשי חכמת השיעור הוא שתהיה מרבע את קוטר הכדור ותכפול המרובע הזה ג' פעמ' ושביעית פעם ויעלה בידך משיחת שטח הכדור | |
|
מנה אותו בשתות הקוטר ויעלה בידך תשבורת גוף הכדור | |
|
כגון כדור אשר קוטרו ז' אמות | |
|
יהיה מרובע הקטר מ"ט אמה | |
|
וכשאתה כופל המספר הזה ג' פעמ' ושביעית פעם יהיה קנ"ד והם מניין שטח אמות הכדור | |
|
מנה אותו בשתות הקוטר והוא אחד ושתות ויהיה ק"פ פחות שליש והוא תשבורת גוף הכדור הזה | |
Segment of a Sphere |
||
|
ומתוך החשבון הזה אתה יכול להבין תשבורת שברי הכדור | |
|
כגון בריכת מים אשר גופה מבפנים מעוגל ופיה עגול ורחבו ז' אמות ועומקו ג' אמות וחצי | |
|
ואתה יודע כי הבריכה הזאת היא חצי כדור ובא ומנה עומקה ברחב פיה אשר הוא קוטר הכדור והנקבץ בידך כפליהו ג' פעמי' ושביעית פעם כאשר עשית בכדור ויעלה ע"ז והוא משיחת שטח הבריכה | |
|
מנה אותו בשתות הקוטר יהיה צ' פחות שתות והוא תשבורת גוף הבריכה אשר היא חצי כדור | |
|
ואלו היה העומק פחות ממחצית הרוחב תהיה יודע שהבריכה הזאת מעוטה מחצי הכדור | |
|
כגון עגולת גוף שעמקה ב' אמות ורוחב עגולת פיה גדר מ' שהוא ו' ושליש בקירוב | |
|
ואתה יודע שהבריכה הזאת אין בה חצי כדור מפני שעומקה פחות ממחצית רוחב פיה | |
|
ואם אתה מוציא את קוטרה כאשר למדת תמצאנו ז' | |
|
ובא ומנה ב' שהוא עומק הבריכה בז' שהוא קוטר הכדור יהיו י"ד | |
|
כפול אותם ג' פעמי' ושביעית יהיו מ"ד והוא משיחת שטח הבריכה | |
|
מנה אותו בשתות הקוטר והוא אחד ושתות יהיו נ"א ושליש והוא תשבורת גוף הבריכה | |
|
ואם היית אומר עומק הבריכה ה' אמות ורוחב פיה גדר מ' | |
|
היות מוצא קוטרה ז' | |
|
ואם אתה מונה העומק בקוטר יהיה ל"ה | |
|
כפליהו ג' פעמ' ושביעית יהיה ק"י והוא משיחת שטח הבריכה | |
|
מנה אותו בשתות הקוטר יהיה קכ"ח ושליש והוא תשבורת רבוע הבריכה וכה"ל | |
Triangular Prism |
||
|
צז מצבה חצויה וקורים למצבה משולשת חצויה מפני שהיא מחצית המרובעת | |
|
מצבה חצויה אשר ראשה ותושבתה משולש נצב הזוית אשר צלעו האחת ג' אמות והשני ד' והשלישית ה' וגובהו י' אמות | |
|
[this figure does not appear in the text] |
||
|
אתה יודע תשבורת המשולש הזה והוא ו' אמות | |
|
מנה אותם בי' שהוא הגובה יהיו ס' אמה והוא תשבורת המצבה חצוית הראש | |
Pentagonal / Hexagonal Prism |
||
|
וכן אלו היה הגולם ראשו ותושבתו שטחים מחומשות או משושתות היא נקראת מצבה מחומשת או משותתת | |
|
תהיה יודע משיחת המחומש או המשותת הזה אשר היא תושבתו או ראשו ותהיה מונה משיחתו במניין הגובה ויעלה בידך תשבורת המצבה ההיא | |
|
| ||
Cylinder |
||
|
ואם יהיה הגולם ראשו ותשברתו שטחים עגולים וכל גופו עולה בעגול עם הראש | |
|
ואתה מונה תשבורת התמונה הזאת על הדרך אשר ידעת התמונות הראשונות | |
|
אם אתה מרבע העגול שהוא התושבת או הראש ואתה מונה מרובעו במניין הגובה יעלה בידך תשבורת התמונה [22]הזאת והיא נקראת מצבה עגולה | |
|
ואני נותן לך דמיון מזה מצבה עגולה אשר ראשה וכן תושבתה שטח עגול אשר קוטרו י' אמות וגובה המצבה י"ד | |
|
||
|
ואתה יכול להגיע אל תשבורת המצבה הזאת אם אתה מונה את קוטר העגול שהוא י' אמות במנין עצמם ויהיו ק' אמות ומנה ק' בי"ד שהם אמות הגובה ויהיו אלף ות' אמות וזה הוא תשבורת התמונה הזאת אלו היתה מרובעת | |
|
הוצא מהמספר הזה שבעיתו וחצי שבעיתו והם ג' מאות אמה ונשאר בידך אלף ומאה אמה והוא תשבורת המצבה העגולה | |
|
| ||
|
וכן אלו היית מוציא מן ק' אמה שהוא רביע התושבת שביעיתו ומחצית שבעיתו והוא כ' אמה וג' חלקי' מז' חלקי' באמה וישאר בידך ע"ח אמה וג' חלקי' מז' באמה והם משיחת העגול אשר היא תושבת המצבה | |
|
| ||
|
מנה אותה בגובה אשר הוא י"ד יעלה המניין לאלף ומאה אמה והוא תשבורת המצבה הזאת העגולה | |
|
| ||
|
ונראה לך שדרך כל המין הזה נוהג מנהג אחד ואם אתה יודע מספר תשבורת מזה התושבת מן התושבת או הראש מאיזו תמונה הייתה ותמנה תשברתה במנין הגובה י' אתה מוצא תשבורת הגוף ההוא ואין שם חילוף | |
Questions about Rectangles |
שאלות במרובע ארוך | |
| Figure Problem - Diagonal - Rectangle | ||
|
צח מרובע ארוך שיש בצלעו האחד ח' אמות ובצלעו השנית ו' כמה הוא אלכסונו | |
|
|
||
|
תשובה מנה ח' שהוא צלע האחת בו' שהוא הצלע השנית ויהיה רבועם מ"ח | |
|
וכפול המרובע הזה והם צ"ו | |
|
ואם תוסיף עליו מרובע ב' אשר היא תוספת הצלע האחת אל השנית יהיה הכל ק' | |
|
וגדר ק' הוא י' והוא אלכסון מן המרובע הזה | |
|
| ||
|
וכללו של ענין שכל מרובע ארוך אם אתה מוסיף רבוע העודף אשר בן שתי צלעיו על כפל תשברתו יהיו אלה שניהם שוים למרובע אלכסונו | |
|
| ||
| Figure Problem - Side; Area - Rectangle | ||
|
צט שאלה אחרת מרובע ארוך אשר באלכסונו י' אמה וארכו מוסיף על רחבו ב' אמות כמה הוא ארכו וכמה הוא רחבו וכמה תשברתו | |
|
|
||
|
תשובה אתה יודע כי מרובע האלכסון הוא ק' | |
|
הוצא ממנו רבוע עדף האורך על הרחב אשר הוא ב' וריבועו ד' ישאר בידך מהם צ"ו | |
|
חלק אותם לשנים ויהיו מ"ח והוא תשבורת המרובע | |
|
| ||
|
ואם תרצה לדעת צלעותיו אשר האחד מוספת על השנית ב' בא וחלק העודף הזה לשנים ויהיה א' וריבועו א' | |
|
הוסיפנו על התשבורת ויהיה מ"ט | |
|
וגדר המניין הזה הוא ז' | |
|
ואם תוסיף עליו אחת שהוא מחצית העודף יהיה ח' והוא קו האורך | |
|
| ||
|
ואם תפחות ממנו א' ישאר ו' והוא קו הרוחב | |
|
ומנין ח' בו' הוא מ"ח והוא התשבורת | |
|
ואם יאמר מרובע בתשברתו מ"ח וקו ארכו עם קו רחבו שניהם יחד י"ד כמה הוא ארכו וכמה הוא רחבו | |
|
ולתשובת השאלה הזאת תקח מחצית י"ד והוא ז' | |
|
ותרבע אותו ויהיה מ"ט | |
|
הוצא מהם מ"ח הוא התשבורת וישאר בידך א' | |
|
וגדר הא' הוא א' | |
|
אם תוסיפנו על ז' יהיה ח' והוא אורך המרובע | |
|
| ||
|
ואם תפחות האחד מן הז' ישאר בידך ו' והוא רוחב המרובע | |
|
והוי יודע כי אם ישאל בענין זה שאלה אשר יהיה בה התשבורת עודף על מרובע מחצית הצלעות כי שאלה זאת שקר וכזב | |
|
כגון האומר לך מרובע שתשברתו מ"ח וקו ארכו עם רחבו י"ג | |
|
טעות הוא בידו [23]או מנסה הוא לך ואינך צריך להשיב | |
Square |
||
| Figure Problem - Diagonal - Square | ||
|
ק שאלה מרובע השוה ונצב הצ' הזוית שארכו י' ורחבו י' כמה הוא אורך אלכסונו | |
|
תשובה האלכסון הזה הוא גדר מאתים כי מרובע האלכסון ימצא ברבועו מאתי' | |
|
מפני שכל מרובע נצב הזוויות רבוע אלכסונו כפלים מהמרובע ההוא | |
|
ומפני זה אמרנו על האלכסון הזה שהוא גדר מאתיי' אשר הוא כפלים מרובע י' על י' | |
| Figure Problem - Side - Square | ||
|
ואם יאמר מרובע שאלכסונו גדר מאתים כמה צלעו | |
|
חלק מרובע האלכסון לשנים ויהיה ק' האחד | |
|
ואמור גדר ק' הוא הצלע המרובע והוא י' | |
|
| ||
|
והאלכסון הזה י"ד ושביעית בקרוב | |
| Figure Problem - Side; Area - Square | ||
|
קא שאלה אחרת מרובע שוה שהוצאת מן מניין תשברתו מניין צלעותיו הארבעה ונשאר בידך מהתשבורת כ"א אמה כמה הוא התשבורת וכמה מניין כל צלע וצלע מהמרובע | |
|
תשובה חלק מנין הצלעות שהוא ד' לשנים | |
|
ומנה השנים בעצמם כלומר רבעם יהיו ד' | |
|
הוסף המניין הזה על המניין המסודר לך הנשאר לך מן המרובע ויהיה הכל כ"ה | |
|
ודע גדר כ"ה והוא ה' | |
|
ותוסיף עליו חצי מניין הצלעות והוא ב' ויהיה הכל ז' והוא צלע המרובע | |
|
| ||
|
ותשברתו מ"ט | |
|
ואם יאמר מרובע אשר הוספת מניין כל ד' צלעותיו על מניין תשברתו ויהיה הכל ע"ז כמה הוא המרובע הזה | |
|
ואתה בשאלה הזאת קח מניין חצי הצלעות והוא ב' | |
|
ותמנה אותו בעצמו ויהיו ד' | |
|
והוסיפנו על המניין שמסר לך והוא ע"ז ויהיו פ"א | |
|
וקח גדר המספר הזה והוא ט' | |
|
הוצא ממנו חצי מספר הצלעות שהוספת וישארו בידך ז' והוא צלע המרובע | |
|
| ||
|
ותשברתו מ"ט | |
| Figure Problem - Side - Square | ||
|
קב שאלה שלישית מרובע השלכת תשברתו ממניין ד' צלעותיו ונשאר בידך ג' | |
|
חלק מניין הצלעות על ב' וחציים הוא ב' | |
|
וריבועם הוא ד' | |
|
הוצא מהם השלשה שנשארו בידך וישאר א' | |
|
אשר גדרו א' | |
|
פחות אותו ממחצית הצלעות וישאר אחד והוא צלע המרובע | |
|
| ||
|
או הוסף עליו גדר האחד שנשאר בידך על מחצית הצלעות יהיו ג' ויהיו גם הם צלע המרובע | |
|
כי יכול יהיה א' הגדר ויכול יהיה ג' כי שני חשבונות לשאלה הזאת | |
Area |
||
Circle |
||
|
קג אם תרצה לדעת תשבורת העגול התמים אם תדע אלכסונו והוא הקוטר | |
|
תכפול אותו ג' פעמי' ושבעית פעם והוא יהיה אורך הקו הסובב | |
|
ואחר כן הוי מרבע מחצית הקוטר במחצית הקו הסובב והוא יהיה תשבורת העגול | |
|
והדמיון לענין הזה עגולה שהקוטר שלה י"ד | |
|
|
||
|
ואתה כופל אותו ג' פעמ' ושביעית ויהיה מ"ד והוא מדת הקו הסובב | |
|
ואם אתה מונה מחצית הקוטר והוא ז' במחצית הקו הסובב והוא כ"ב יהיה המניין קנ"ד אמות והוא תשבורת העגול | |
Triangle |
||
|
קד הרי שיש לפניך אי' משולש כזה ותרצה לידע מדת שבריו | |
|
|
||
|
תרבה חצי צלע העליון על כל העמוד וכן יהיו מדת שבריו | |
|
המשל בזה הרי שהיה צלע העליון י' אמות והעמוד שלו י"ב אמות | |
|
תקח [24]חצי צלע העליון שהוא ה' ותרבה אותו על כל העמוד שהוא י"ב ואמור ה' פעמ' י"ב ס' וכן יבא | |
Square |
||
|
קה המרובע שהוא שוה הצלעות וכל זוויותיו נצבות ותרצה לידע תשברתו | |
|
תמדוד אחד מצלעיו ותרבה אותו בעצמו וכפי מה שיעלה כן יהיה | |
|
המשל בזה מרובע אחד שכל אחד מצלעותיו ד' וזוויותיו נצבות | |
|
|
||
|
תמדוד אחד מצלעותיו שהוא ד' ותרבה אותו בעצמו ואמור ד' פעמ' ד' י"ו וכן יהיה מניין תשברתו וכה"ל | |
Triangle whose base is an arc - Sector |
||
|
קו משולש אשר תושבתו עקמימות ב"ג וב' צלעיו הם קוים ישרים והם כצורה אשר אני מצייר לך ב' קוים א"ב וא"ג והתושבת קו עקום אשר עליו בד"ג | |
|
ואם אתה עושה כצורה הזאת קו כגון קו ב"ג יהיה לך משולש ישר אשר עליו אב"ג וצורת העקום אשר עליה בד"ג והיא שבר עגולה | |
|
|
||
|
ואתה מרבע כל אחד מהם לבד ותאסוף שני המרובעים ויהיו תשבורת הצורה | |
Rectangle |
||
|
קז המרובע ארוך שלא תהיינה צלעותיו שוות זו לזו אבל הנכוחים לבד הם שוות דהיינו שיהיו שני הארוכים שוים ושני הרחבים שוים וכל הזוויות הם נצבות ותרצה לידע תשברתו | |
|
תרבה צלע האורך עם צלע הרוחב וכאשר יעלה כן יהיה תשברתו | |
|
כגון מרובע ארוך ששתי צלעותיו האורך כל אחד מהם ט' אמות ושתי צלעות הרוחב כל אחד מהם ה' אמות כזה | |
|
|
||
|
תרבה האורך עם הרוחב ואמור ה' ט' הם מ"ה וכן יהיה מניין תשברתו וכה"ל | |
Rhombus |
||
|
קח המרובע שהוא שוה הצלעות ואינו נצב הזוויות והוא הנקרא מרובע המעויין כגון מרובע שיש בכל צלעותיו י' אמות ואינו נצב הזוויות ואתה מוצא אלכסונותיו שאינן שוים כגון שיהיה האלכסון האחד י"ב אמות ואלכסון השני י"ו אמות | |
|
|
||
|
תמנה האלכסון האחד במחצית האלכסון השני | |
|
ואמור י"ו פעמי' ו' הם צ"ו וכן יהיה מנין תשברתו | |
|
או אמור י"ב פעמ' ח' צ"ו וכן יהיה מניין תשברתו וזו היא הצורה | |
Equilateral Triangle |
||
|
קט משולש שוה הצלעות ותרצה לדעת תשברתו | |
|
|
||
|
דע כי תשברתו הוא שלישית מרובע צלעו ועשירית מרובע הצלע | |
|
כגון משולש שכל אחד מצלעיו ט"ו | |
|
תרבה אחת מצלעיו ואמור ט"ו פעמי' ט"ו הם רכ"ה | |
|
קח שלישית רכ"ה שהוא ע"ה ועשירית רכ"ה שהוא כ"ב וחצי ויהיה הכל צ"ז וחצי וכן יהיה תשבורת זה המשולש וכה"ל וזאת היא הצורה | |
|
| ||
|
ואם תרצה לדעת תשבורת המשולש בעניין אחר | |
|
תרבע העמוד [25]היוצא בו במחצית התושבת או תרבע התושבת במחצית העמוד | |
|
כגון משולש שכל אחד מצלעותיו ט"ו ועמודו י"ג כזה | |
|
תרבה העמוד שהוא י"ג במחצית התושבת שהוא ז' וחצי ואמור י"ג פעמ' ז' וחצי הם צ"ז וחצי וכן יהיה תשבורת המשולש | |
|
או תרבע התושבת שהוא ט"ו במחצית העמוד שהוא ו' וחצי ואמור ט"ו פעמ' ו' וחצי צ"ז וחצי וכן יהיה תשברתו וכה"ל | |
Equilateral Triangle |
||
| Figure Problem - height; Area - Equilateral Triangle | ||
|
קי ואם תרצה לדעת שבור העמוד מן המשולש שהוא שוה הצלעות עשה כענין זה כגון משולש שכל אחד מצלעותיו י' כזה | |
|
|
||
|
תרבה הצלע האחד בעצמו ואמור י' פעמ' י' הם ק' | |
|
ותרבה חצי התושבת שהוא ה' בעצמו ואמור ה' פעמ' ה' כ"ה | |
|
ותפחות אותם מק' ישארו ע"ה | |
|
ותמצא הגדר של ע"ה והנו ח' וב' שלישי בקירוב והוא יהיה מספר העמוד | |
|
| ||
|
ואם תרצה לדעת שברי זה המשולש | |
|
תרבה כל העמוד עם חצי התושבת שהוא ה' | |
|
או תרבה כל התושבת במחצית העמוד | |
| Figure Problem - height; Area; Side - Equilateral Triangle | ||
|
קיא ואם תרצה לדעת שיעור העמוד בעניין אחר מן המשולש שהוא שוה הצלעות | |
|
תפחות לעולם מן הצלע שלמשולש שוה הצלעות ב' חלקי' מט"ו והוא יהיה מספר העמוד | |
|
כגון משולש שכל אחד מצלעותיו [ט"ו]
הוי יודע כי עמודו י"ג | |
|
תרבה י"ג שהוא העמוד בז' וחצי שהוא חצי התושבת | |
|
או כל התושבת שהוא ט"ו בו' וחצי שהוא חצי השיעור מן העמוד | |
|
ואם תדע העמוד מן המשולש השוה הצלעות ותרצה לדעת הצלע מן העמוד ההוא | |
|
תרבע העמוד בעצמו והוסיף על זה המרובע שלישיתו ותידע כמה הוא גדר הכל והוא יהיה מדת כל צלע מצלעי המשולש שוה הצלעות | |
|
כגון משולש שהעמוד שלו הוא י"ג ותרצה לידע מידת הצלע | |
|
תרבה העמוד בעצמו ואמור י"ג פעמ' י"ג קס"ט | |
|
הוסף עליהם שלישית קס"ט שהם נ"ו ושליש יהיו רכ"ה ושליש | |
|
תמצא הגדר של רכ"ה ושליש שהם ט"ו בקירוב והוא יהיה מספר הצלע | |
|
| ||
Scalene Triangle |
||
| Figure Problem - Side - Scalene Triangle | ||
|
קיב אם יאמר לך אדם משולש מתחלף עמודו י"ב והצלע האחר המקפת את ראשו י"ג והשנית ט"ו כמה תהיה התושבת | |
|
והתשובה בשאלה הזאת תרבע הצלע האחת כגון י"ג שריבועה קס"ט | |
|
ותוציא ממנו מרובע העמוד והוא קמ"ד ישאר כ"ה שגדרו ה' והוא המעמד האחד | |
|
ושוב ורבע את הצלע השנית ומרובעה רכ"ה | |
|
הוצא ממנה מרובע העמוד ישאר פ"א וגדרו ט' והוא המעמד השני | |
|
וב' המעמדי' האלה הם י"ד והוא אורך התושבת | |
|
| ||
| Figure Problem - height - Scalene Triangle | ||
|
ואם יאמר לך משולש מתחלף בתשברתו עם העמוד צ"ו ותושבת העמוד י"ד כמה הוא העמוד | |
|
תשובה קח מחצית התושבת והוסף אחד לעולם וחלק עליה את המספר אשר בידך ותמצא העמוד | |
|
כאילו היית מחלק בשאלה הזאת התושבת לשנים ומחציתה הוסף עליה אחד יהיו ח' חלק עליהם צ"ו יהיה י"ב והוא אורך העמוד | |
Isosceles Triangle |
||
| Figure Problem - Area; height; Side - Isosceles Triangle | ||
|
קיג משולש שוה השוקים והוא משולש ששוקיו שוים והתושבת אינו שוה עמהם [26]כגון זה המשולש שכל אחד משוקיו ט"ו והתושבת היא י"ח | |
|
|
||
|
נדע מספר העמוד תחלה ונעשה בענין זה | |
|
נרבע אחד מן השוקים בעצמו ואמור ט"ו פעמי' ט"ו הם רכ"ה | |
|
ונפחות מרכ"ה מרובע חצי התושבת וחצי התושבת הוא ט' ומרובעו פ"א ונפחות אותם מרכ"ה ישארו קמ"ד | |
|
ותמצא השורש מקמ"ד והוא י"ב וכן יהיה מספר העמוד הזה י"ב | |
|
| ||
|
ואם תרצה לדעת תשבורת המשולש הזה | |
|
תרבה כל העמוד שהוא י"ב על חצי התושבת שהוא ט' ואמור ט' פעמ' י"ב הוא ק"ח והוא תשבורת זה המשולש | |
|
או תרבה כל התושבת שהוא י"ח על חצי העמוד שהוא ו' ואמ' ו' פעמ' י"ח הוא ק"ח והוא יהיה תשברתו וכה"ל | |
|
ואם תדע מדת העמוד ומדת התושבת ממשולש שוה השוקים ותרצה לדעת מדת כל אחד מהשוקים כגון זה המשולש שעמודו י"ב ותושבתו י"ח | |
|
תרבע העמוד בעצמו שהוא י"ב ואמור י"ב פעמ' י"ב הם קמ"ד | |
|
הוסף עליהם מרובע חצי התושבת שהוא ט' שיבא פ"א וקבצם יהיו רכ"ה | |
|
תמצא השורש מרכ"ה והוא ט"ו וכן יהיה מדת כל אחד מהשוקיים וכה"ל | |
|
| ||
Area |
||
Scalene Triangle |
||
|
קיד משולש מתחלף הצלעות כגון משולש אב"ג שצלע א"ב י"ג וצלע ב"ג י"ד וצלע א"ג ט"ו | |
|
|
||
|
וזה המשולש לא נוכל לדעת תשברתו אלא מן העמוד | |
| [Although later on (problem 116) a general formula is given for finding the area of any triangle according to its three sides without needing to know the height] | ||
|
כי כל משולש בין שוה הצלעות בין מתחלף הצלעות תשברתו הוא ברבוע העמוד על חצי התושבת או בריבוע כל התושבת בחצי העמוד | |
|
| ||
|
ולכן נצטרך להוציא העמוד ולהגביל מעמדו בתושבת | |
|
כי העמוד במשולש הזה אינו עומד במחצית התושבת אבל נוטה ממחצית התושבת אל צד אחד | |
|
והצד הארוך מגבול מעמדו אנו קוראים לו מעמד ארוך והצד השני מעמד קצר | |
|
ואם נרצה להוציא המעמד הארוך | |
|
נקח מרובע הצלע משתי הצלעות אשר העמוד יוצא מבניהם והוא צלע א"ג שהוא ט"ו ומרבעו רכ"ה | |
|
ונחבר המרובע הזה אל מרובע התושבת שהוא י"ד ומרובעו קצ"ו ויהיו שני המרובעים תכ"א | |
|
נוציא מהם מרובע צלע הקצר שהוא צלע א"ב שהוא י"ג ומרובעו קס"ט וישארו רנ"ב | |
|
נחלק רנ"ב לשנים ויהיה המחצית קכ"ו | |
|
נחלק קכ"ו על התושבת שהוא י"ד יהיו ט' והוא מרחק גבול מעמד העמוד מן הצלע הארוך הרי שקו ג"ד הוא ט' | |
|
| ||
|
ואם נרצה לדעת המעמד הקצר | |
|
נקח מרובע הצלע הקצר שהוא י"ג ומרובעו קס"ט | |
|
עם מרובע התושבת שהוא קצ"ו ויהיו שני המרובעי' שס"ה | |
|
נוציא מהם רכ"ה שהוא מרובע קו א"ג שהוא הצלע הארוך שהוא ט"ו ישארו ק"מ | |
|
חלקם לשנים ויהיה המחצית ע' | |
|
נחלקם על התושבת שהוא י"ד יהיו ה' וכן מרחק מעמד העמוד מן הצלע הקצר | |
|
| ||
|
ואם תרצה לדעת אורך זה העמוד | |
|
נרבע הצלע ונוציא ממרובעו מרובע המעמד הדבק בו ונקח גדר הנשאר והוא יהיה אורך העמוד | |
|
| ||
|
המשל בזה נרבע הצלע הקצר שהוא י"ג בזה המשולש יהיו קס"ט | |
|
נפחות מקס"ט מרובע המעמד הקצר הדבק בו דהיינו קו ד"ב שהוא ה' ומרובעו כ"ה ישארו קמ"ד | |
|
וגדר קמ"ד הוא י"ב [27]הרי שמספר העמוד הוא י"ב | |
|
| ||
|
וכן אם נרבע הצלע הארוך שהוא ט"ו ומרובעו רכ"ה | |
|
ונפחות ממנו מרובע המעמד הארוך דהיינו קו ג"ד שהוא ט' ומרובעו פ"א ישארו קמ"ד כמו שנשאר מן הצלע הקצר | |
|
וגדר קמ"ד הוא י"ב והוא אורך העמוד | |
|
| ||
|
ואם תרצה לדעת תשבורת זה המשולש | |
|
תרבה כל העמוד שהוא י"ב בחצי התושבת שהוא ז' שיבואו פ"ד והוא תשברתו | |
|
או תרבה כל התושבת שהוא י"ד בחצי העמוד שהוא ו' ויהיו פ"ד והוא תשברתו וכה"ל וזאת היא צורתה | |
Right Triangle |
||
|
קטו המשולש הזה נקרא משולש נצב הזויות ואם תרצה לדעת תשברתו | |
|
תרבה הצלע האחד מן הצלעות המקיפות בזוית הנצבה בחצי הצלע האחרת | |
|
כגון אם היה קו ב"א י' וקו ב"ג ג"כ י' | |
|
|
||
|
אמור י' פעמ' ה' הם נ' והוא תשברתו | |
|
כי אתה רואה שהוא חצי מרובע שוה הצלעות נצב הזוויות | |
|
ואם היה מרובע שלם היינו אומרי' י' פעמ' י' הם ק' | |
|
ועכשיו שהוא חצי מרובע נאמר י' פעמ' ה' הם נ' וכן לכל חשבון כזה | |
|
| ||
Heron's Theorem |
||
|
קיו כלל גדול לדעת תשבורת המשולש שאינך צריך בו אל הוצאת העמוד והחשבון הזה נקרא חשבון המותרות | |
|
והוא שתדע מחצית כל אחד מצלעי המשולש ותקבץ כל המחציות האלה | |
|
ותדע מותר כללם על כל צלע וצלע ותשמור המותרות האלה | |
|
ותמנה אחד מהם בשיני והמספר הנקבץ מנה אותו בשלישי במספר | |
|
ואשר יכנס בידך מהחשבון הזה מנה אותו בכל כלל המחציות אשר קבצת | |
|
ויהיה המספר הזה מרובע תשבורת המשולש | |
|
ואם תוציא גדר המספר הזה תמצא התשבורת | |
|
| ||
|
המשל בזה משולש שצלעו האחד י' והשני ח' והשלישי ו' | |
|
|
||
|
ואם אתה מקבץ מחצית ג' הצלעות האלה יהיה המספר י"ב | |
|
ומותר י"ב על הצלע האחד ב' ועל השני ד' ועל השלישי ו' | |
|
ואתה בוא ומנה ב' שהוא מותר הצלע האחד בד' שהוא מותר הצלע השני יהיו ח' | |
|
בוא ומנה אלו הח' בו' שהוא מותר הצלע השלישי ויהיו מ"ח | |
|
שוב ומנה מ"ח בי"ב שהוא כלל המחציות ויהיו תקע"ו ותקע"ו הוא מרובע התשבורת | |
|
ואם תמצא הגדר של תקע"ו יהיו כ"ד והוא יהיה תשבורת זה המשולש וכה"ל | |
|
| ||
Parallelogram |
||
|
קיז מרובע ששני אורכיו שוים וכל אחד מהם כ"ה ושני רחביו שוים וכל אחד מהם ט"ו ואין זוויותיו נצבות כגון זה | |
|
|
||
|
כשנבוא לרבעו נמדוד בו אלכסון א"ג והיה כ' אמה והוא החולק הזה המרובע לב' משולשים שוים ואין אנו יכולין לדעת שברי זה המרובע אלא מתוך ריבוע ב' המשולשים האלה | |
|
ומפני זה אם אנו מוציאים את העמוד באחד מהם נדע תשבורת המשולש ההוא ונכפול אותו ונמצא תשבורת שני המשולשים דהיינו תשבורת כל זה המרובע | |
|
ואם תוציא עמוד [28]במשולש אב"ג על צלע א"ב יהיה העמוד י"ב ותשבורת המשולש ק"נ וכפל ק"נ הוא ש' והוא תשבורת המרובע הזה | |
|
ומי שלא היה מבין זה הדרך והיה מרבע האורך עם הרוחב כשאר המרובעים שהם על זוויות נצבות והיה אומ' כ"ה פעמ' ט"ו היו עולים שע"ה ראה כמה טעות בין שני החשבונות המרובע עודף | |
| Additional segment: the differences between the square and its inner circle and the inner square of the inner circle | ||
|
המרובע עודף על העגול שבתוכו העגול עודף על המרובע שבתוכו | |
|
||
Trapezoid |
||
Isosceles Trapezoid |
||
|
קיח קטומת הראש כגון זאת הצורה שיש לה ד' צלעות ועליהם אבג"ד וצלע א"ב וג"ד הם שוות וכל אחד מהם י"ג | |
|
והצורה הזאת נקראת קטומת הראש שוה | |
|
וצלע א"ג שהיא הקצרה משתי צלעות הנכוחייות נקראת ראש הקטומה | |
|
וצלע ב"ד הארוכה נקראת תושבת הקטומה | |
|
|
||
|
והרוצה למדוד הצורה הזאת יוציא עמודה ראשונה | |
|
ודרך הוצאתו הוא שיפחות ראש הקטומה משרשה מתושבתה | |
|
והעודף ביניהם יחלק אותו לשנים | |
|
וידע מרובע המחצית | |
|
ויפחות מהמרובע הזה מרובע אחת מן הצלעות השוות | |
|
והנשאר ממרובע הצלע ידע שרשו והוא יהיה מספר העמוד | |
|
ובצורה הזאת נפחות מנין ח' שהוא ראש הקטומה מן י"ח שהוא התושבת ישאר י' | |
|
והמחצית מי' הוא ה' והוא יהיה ודאי חלק העודף האחד מנקודת ה' קו ב"ה ומנקודת ז' קו ד"ז | |
|
ונוציא קו מא' אל ה' ומן ג' אל ז' ובידוע שכל קו מהם הוא עמוד על תושבת ב"ד | |
|
ויהיה משולש אה"ב נצב הזוית ומיתר הזוית הזאת הוא קו א"ב | |
|
ובידוע כי מרובע המיתר הזה שהוא י"ג שוה למרובע ב"ה שהוא אשר ידענו שהוא ה' ולמרובע א"ה שאנו רוצים לדעתו | |
|
ואם נפחות מרובע ה' שהוא כ"ה ממרובע י"ג שהוא קס"ט ישאר קמ"ד | |
|
ובידוע שגדר קמ"ד והוא י"ב יהיה מדת העמוד | |
|
| ||
|
ואם תרצה לדעת תשבורת זאת הצורה | |
|
תכניס הראש עם התושבת ויהיו כ"ו | |
|
קח מחציתם והם י"ג | |
|
ומנה אותם בי"ב שהוא מדת העמוד יהיו קנ"ו והוא תשברתו | |
|
| ||
|
ואם תרצה להאריך למעלה שני צלעי הקטומה שהם קו א"ב וג"ד עד שיפגשו אל נקודת ז' כמו שתראה בצורה ותהיה הצורה דומה למשולש | |
|
|
||
|
ותרצה לדעת אורך הקוים העולים למעלה והם קוי א"ז וג"ז | |
|
תדע עודף התושבת על הראש שהוא י' בצורה הזאת ותשמור אותו עמך | |
|
ושוב ותראה הראש שהוא ח' בכל הצלע [29]שהוא י"ג ויהיה הכל ק"ד | |
|
חלקם על י' אשר הוא העודף אשר שמרת ותהיה החלוקה י' ושני חומשי' והוא אורך קו א"ז וכמו כן קו ג"ז העולים עד הנקודה | |
|
| ||
|
או תעשה על זה הדרך שתדע ערך ח' אמות שהוא אורך הראש מי' שהוא עודף התושבת על הראש | |
|
וערך ח' אל י' הוא ד' חמשי' דהיינו א' פחות חומש | |
|
וכערך ח' אל י' כן יהיה ערך הקו העולה עד נקודת הז' אל י"ג שהוא הצלע | |
|
| ||
|
דהיינו שיהיו י"ג פחות י"ג חומשי' שהם י' וב' חמשים וכה"ל | |
Trapezoid |
||
|
קיט רבוע הקטומה הזאת הוא בריבוע אחד מעמודה במחצית הראש והתושבת יחד | |
|
|
||
|
והוא שתהיה מקבץ בצורה הזאת ח' שהוא אורך הראש כ"ב שהוא התושבת ויהיה הכל ל' | |
|
קח מחציתו והוא ט"ו ומנה אותו בי"ב שהוא אורך העמוד ויהיה הכל ק"פ והוא יהיה תשבורת הקטומה הזאת וזאת היא הצורה | |
Right Trapezoid |
||
|
קכ רבוע הקטומה הזאת | |
|
|
||
|
הוא באסיפת ראשה אל תושבתה ותקח מחצית' וימנה בעמוד והוא יהיה תשברתה | |
|
ואם תאסוף בצורה הזאת ראשה אל תושבתה יהיה כללם כ"ח | |
|
קח מחציתם שהוא י"ד ותרבה אותו בט' שהוא אורך העמוד יהיו קכ"ו והוא תשבורת הקטומה הזאת | |
Trapezoid |
||
|
קכא תשבורת הקטומה הזאת | |
|
|
||
|
הוא ברבוע העמוד בחצי הראש וחצי התושבת | |
|
וחצי הראש וחצי התושבת הם י"ז וחצי | |
|
תרבה אותם על י"ב שהוא העמוד יהיו ר"י והוא תשברתו וכה"ל | |
| Figure Problem - Quadrangle whose two sides are equal to its diagonal | ||
|
קכב מרובע שני קוויה כמרובע אלכסונה | |
|
||
Arc |
||
Half a Circle; Arc |
||
|
קכג צורת חצי עגול אם תרצה לדעת תשבורת הצורה הזאת | |
|
תרבע חצי המיתר שהוא חצי קוטר העגול בחצי הקו העקום הנקרא קשת ותמצא התשבורת | |
|
ואם תרצה לדעת מדת הקשת תכפול חצי המיתר שהוא ד' ג' פעמ' ושביעי' פעם [30]ויהיו י"ב אמות וד' שבעיות וכן יהיה מדת הקשת ר"ל הקו הסובב עקמומית זה החצי העגול | |
|
|
||
|
ואם תרצה לדעת שברי זה החצי העגול תרבע כאשר אמרנו חצי המיתר בחצי הקו הסובב דהיינו שתאמ' ד' פעמ' ו' וב' שבעיות יהיו כ"ה ושביעית וכן יהיה תשבורת חצי העגול וכה"ל | |
|
או תעשה על דרך זה תרבע את המיתר בעצמו ותוציא מזה המרובע שביעיתו וחצי שביעיתו וקח מחצית הנשאר והוא יהיה תשבורת חצי העגול | |
|
ובצורה הזאת אשר עשינו תרבע המיתר שהוא ח' בעצמו יהיו ס"ד | |
|
הוצא ממנו שבעיתו וחצי שביעיתו שהם י"ג אמות וה' שבעיות ישארו נ' אמות וב' שביעי אמה | |
|
קח מחציתם שהם כ"ה ושביעית והוא יהיה תשבורת חצי העגול | |
|
| ||
Arc, smaller than half a circle |
||
|
קכד קשת פחות מחצי עגול הדמיון לצורה המעוטה מחצי העגולה היא צורת קשת אב"ג ומיתרה א"ג ואורכו ח' וחיצה ד"ב ואורכו ב' | |
|
|
||
|
ואי אתה יכול להגיע לתשבורת הצורה הזאת אם אינך יודע כל קוטר העגול שזה הקשת נקטם ממנו | |
|
ותוכל לדעת קוטר העגול בעניין זה תרבע מחצית המיתר ותחלק מרובעו על החץ והוסף מדת החץ עליו ותדע סך הכל והוא יהיה אורך כל הקוטר | |
|
ומצורה הזאת אשר עשינו | |
|
תרבע מחצית המיתר שהוא ד' בעצמו יהיו י"ו | |
|
וחלק אותו על החץ שהוא ב' יהיו ח' והוא האורך הנשאר מן הקוטר | |
|
הוסף עליהם ב' שהוא החץ יהיו י' | |
|
הרי שכל הקוטר מן העגול שזה הקשת נקטם ממנו הוא י' וכה"ל | |
|
ולראות העניין באר הטב הוי משלים העגולה הזאת כלה | |
|
והוצא קו ב"ד עד שיגיע אל עקמימות העגולה מן הצד השני כגון קו בד"ז | |
|
ואם תרצה לידע תשבורת הצורה הזאת | |
|
חלק קו ב"ז לב' חלקי' שוים על נקודת ח' ותהיה הנקודה הזאת ציר העגולה | |
|
ותרצה והוצא ממנה ב' קווים אל נקודת א' ואל נקודת ג' והם קוי ח"א וח"ג | |
|
|
||
|
ואם אתה מרבע קו א"ח שהוא חצי הקוטר העגול בחצי הקשת אשר הוא עוקם א"ג יהיה מנין הזה תשבורת המשולש אשר ב' צלעיו הם ב' קוי א"ח וח"ג | |
|
ותשבורת קשת אב"ג הוצא מן המנין תשבורת המשולש אח"ג וישאר בידך תשבורת הצורה שעליה אבג"ד | |
|
ותשבורת המשולש הזה הוא רבוע קו ח"ד שהוא בצורה הזאת ג' אמות בחצי קו א"ג והוא ד' אמות ורבועם י"ב | |
|
והוא הסך אשר תוציא מרבוע קו א"ח הישר בקו א"ב העקום ויהיה הנשאר תשבורת צורת אבג"ד שהוא צורת הקשת שהוא פחות מחצי עגול | |
|
| ||
|
ומכאן אתה אומ' כי רבוע הקשת שהוא פחות מחצי עגול הוא שתרבע חצי כל הקוטר מן העגול שהקשת נקטם ממנו בחצי הקשת ותשמור אותו | |
|
ואחרי כן תוציא מחצי הקוטר קו החץ אשר לקשת והנשאר מחצי הקוטר מנה אותו במחצית המיתר | |
|
והעולה בידך תפחות אותו מן המנין אשר שמרת בידך והנשאר בידך הוא תשבורת הקשת שהוא [31]פוחת מחצי עגול | |
|
| ||
Arc, greater than half a circle |
||
|
קכה צורת הקשת העודף על חצי העגול והוא קשת אשר עליו אב"ג ומיתרו א"ג ואורכו י"ב | |
|
|
||
|
ואם תרצה לדעת תשבורת הצורה הזאת תוציא קוטר כל העגול שזאת הצורה נקטמת ממנו ותרבה חצי הקוטר בחצי הקשת ותוסיף עליו תשבורת המשולש אשר המיתר הוא תושבתו ויהיה סך הכל תשבורת כל זאת הצורה | |
|
ובצורה הזאת תרבע חצי המיתר שהוא ו' בעצמו שיבואו ל"ו | |
|
חלק אותם על החץ שהוא י"ב ויהיה החלוקה ג' | |
|
הוסיפם על החץ יהיה סך הכל ט"ו והוא אורך כל הקוטר מן העגול שזאת הצורה נקטמת ממנה | |
|
ומחציתו הוא מחצית הקוטר והוא בצורה הזאת קו ב"ז הרי שקו ב"ז הוא ז' וחצי | |
|
מנה אותו במחצית הקשת ומה שיעלה יהיה תשבורת הצורה העקומה אשר יקיפו אותה ב' קווי ג"ז וא"ז עם קשת א'ב'ג' | |
|
הוסף עליהם תשבורת משולש א'ז'ג' והוא המספר הנקבץ מרובע קו ד"ז במחצית קו א"ג וכשתקבץ הכל יהיה תשבורת העקמומית הזאת | |
|
| ||
|
ומכאן אתה למד הרוצה לדעת תשבורת העקום העודף על חצי העגול יהיה מרבע חצי הקוטר בחצי הקשת וישמור המספר | |
|
ואחרי כן יפחות חצי הקוטר מן החץ | |
|
[והנשאר מן החץ] ירבע אותו בחצי המיתר | |
|
והמספר העולה יאספינו אל המספר אשר שמר ויהיה הכל סך תשבורת העקומה העודפת על חצי העגול | |
|
| ||
Divide a Figure |
||
Triangle |
||
|
קכו אם תרצה לחלק המשולש לב' חלקי' שוים וישאר החלק האחד משולש והשני דומה למרובע | |
|
כגון משולש א'ב'ג' אשר ראשו נקודת א' ותושבתו ב"ג ותרצה לחלקו לחצי | |
|
אתה צריך לחלק כל אחד מצלעי א"ב וא"ג לב' חלקי' בענין שיהיה מרובע הצלע כולו כפליים ממרובע הצלע החלק הגדול מחלקיו | |
|
כגון שתהיה חולק קו א"ב לב' חלקים על נקודת ד' וקו א"ג לב' חלקי' על נקודת ה' | |
|
|
||
|
ויהיה מרובע א"ד מחצית מרובע א | |
|
וכן מרובע א"ה מחצית מרובע א"ג | |
|
ותוציא קו מד' על ה' והיה משולש נחלק לב' חלקי' שוים החלק האחד משולש א'ד'ה' וחלק השני נפתל ד'ה'ב'ג' | |
|
ודרך חשבון המרובעים האלה יהיה אם תהיה לוקח מאורך הקו מנקודת א' אשר הוא הראש ה' חלקי' מז' בו פחות חצי עשירית השביעית והכלל המסור לזה הוא שתהיה לוקח מן הקו צ"ט חלקי' מק"מ חלקי' בו | |
|
כאילו היה אורך צלע א"ב ז' אמות | |
|
יהיה קו א"ד מהם ה' אמות פחות חלק אחד מעשרים באמה | |
|
וצלע א"ג היה אורכו י' אמות | |
|
יהיה קו א"ה ז' אמות וחלק אחד מי"ד באמה | |
|
ועל החשבון הזה אתה חולק את שתי הצלעות האלה ותוציא קו מן החלק האחד אל השני ויחלק המשולש לב' חלקים שוים | |
Triangular Field |
||
|
[32]קכז ואם יהיה קרקע המשולש לג' בעלים ואתה צריך לחלקו ביניהם וכל אחד מהבעלים מבקש חלקו נגד צלע אחד מצלעי המשולש | |
|
ויהיה המשולש הזה משולש א'ב'ג' | |
|
ואתה בא וחלק צלע א"ב לב' חלקי' שוים על נקודת ד' | |
|
והוצא קו מד' אל ג' ויחלק המשולש לב' חלקי' שוי' | |
|
|
||
|
ואחר כן מדוד מקו ד"ג אשר הוא קומת המשולש שלישיתו מנגד נקודת ד' אשר על התושבת ויהיה החלק הזה קו ד"ה | |
|
והוצא מנקודת ה' ב' קוים אל נקודת א' ואל נקודת ב' | |
|
ויחלק המשולש לג' חלקים שוים והם משולש ג'ה'א' ומשולש א'ה'ב' ומשולש ג'ה'ב' | |
Rhombus |
||
|
קכח שאלות במעויין הנז' למעלה אשר אחד מאלכסוניו י"ו והשני י"ב כמה הוא צלעו | |
|
תשובה קח מחצית כל אחד מאלכסוניו ורבע אותם וקבץ שני המרובעי' וקח את גדרם ותמצא הצלע | |
|
ופירוש התשובה | |
|
קח מחצית י"ו והוא ח' וריבועו ס"ד | |
|
וקח מחצית י"ב והוא ו' וריבועו ל"ו | |
|
ומניין שני המרובעים האלו הם ק' | |
|
וגדר ק' הם י' והוא צלע המרובע | |
|
| ||
|
ואם יאמר מעויין שתשברתו צ"ו ואלכסון האחד י"ו כמה הוא האלכסון השני | |
|
חלק צ"ו אשר הוא התשבורת על האלכסון אשר ידעת שהוא י"ו ותמצא מחצית האלכסון השני ו' וכפלה י"ב והוא האלכסון השני | |
|
ואם יאמ' מעויין צלעו י' ותשברתו צ"ו כמה אלכסונו | |
|
קח מרובע הצלע והוא ק' הוסף עליו התשבורת ויהיו קצ"ו וגדר המספר הזה הוא י"ד והם שני חציי האלכסונות | |
|
| ||
|
קח מחצית המספר והוא ז' ומרובעו מ"ט | |
|
הוצא ממנו מחצית התשבורת אשר הוא מ"ח וישאר בידך א' | |
|
וגדר הא' הוא א' אם אתה מוסיף אותו על ז' יהיו ח' והוא מחצית האלכסון האחד | |
|
| ||
|
ואם אתה פוחת אותו מן ז' ישאר ששה והוא מחצית האלכסון השני | |
|
| ||
| Figure Problem - Divide a Figure - Triangle | ||
|
קכט שאילה משולש אשר צלעו האחד י"ב אמות והוא צלע א"ב וצלע ב"ג השני י' אמות וצלע א"ג השלישי ט"ו אמות ואתה רוצה לחלקו לב' בעלים לכל אחד חלק שוה | |
|
|
||
|
והנה נשים נקודות ד' אשר הוא המיתר רחוקה מנקודת ב' על צלע א"ב שתי אמות | |
|
וישאר קו א"ד י' אמות | |
|
ויהיה מחצית צלע א"ב על נקודת ז' ויהיה קו א"ז שש אמות כי כן מסרנו צלע א"ב כולו י"ב אמות | |
|
ובין קו א"ז שהוא המחצית ובין קו א"ד ארבע אמות והם שני חמישיות קו א"ד | |
|
ואתה קח מצלע א"ג הנדבק אל א"ד שני חמשיותיו אשר היא הראש על נקודת ה' | |
|
ויהיה קו ג"ה שש אמות כי כל צלע א"ג היה ט"ו אמה | |
|
והוצא המחצה מנקודת ד' עד נקודת ה' | |
|
ויהיה המשולש הזה נחלק לשני חלקי' שוים החלק האחד משולש ד'א'ה' והחלק השני נפתל ד"ה ג"ב | |
|
ואילו היה המיצר אשר הוא נקודת ד' נופל לצד אחד עד שיהיה א"ד שתי אמות לא היית מוצא המחצה אל הצלע א"ג אבל אל צלע ב"ג | |
|
והיית לוקח מצלע ב"ג אשר הוא י' אמות שני חמשיותיו על נקודת ה' מנגד נקודת ג' | |
|
ויהיה קו ג"ה [33]בצלע ב"ג ד' אמות | |
|
ותוציא המחצה מנקודת ד' אל נקודת ה' כאשר אתה רואה בצורה הזאת | |
|
כי המחצה בדמיון הזה היא יוצאה לעולם אל החלק הארוך | |
|
אם יהיה הארוך ב"ד אתה מוציא המחצה אל צלע ב"ג | |
|
ואם יהיה הארוך א"ד אתה מוציא המחצה אל צלע א"ג | |
|
ואתה מתחיל למדוד את שתי החמשיות מנקודת ג' אשר היא הראש ואל הנקודה הזאת הייתה המחצה יוצאה אלו היה המיצר נופל על מחצית צלע א"ב ומדת מרחק המיצר מן המחצית אתה מתרחק מנקודת ג' אשר הוא הראש | |
|
ואתה יכול להבין את העניין הזה אם לא היית מדמה אותו במספר אלא שזכרתי המספר כדי להקל על הלומד ולהקריב העניין ללב המלמד | |
Area |
||
Polygons |
||
|
קל שאלה במדידת הצורות אשר צלעותיו עודפות על ארבע הצלעות והצורות האלה הם על תמונות רבות מהם מחומשות ומהם משותתות ומשובעות ולמעלה מהם יש מהם שצלעותם וזוויותם שוות ויש שלא יהיו שוות אלא מתחלפות | |
|
ואני נותן לך כלל ראשונה במדידת הצורות אשר צלעותן וזוויותן שוות | |
|
והכלל הזה הוא כל תבנית וכל צורה אשר אין לה קוים ישרים אם אתה מעגיל בתוכה עגול שיהיה מושש לצלעיה ידוע הוא כי רבוע קוטר חצי העגול הזה במחצית כל צלעי הצורה ההיא א"ו היא תשברתה | |
|
וכל צורה מן המרבע ולמעלה אשר צלעיה וזוויותיה שוות זו לזו אתה יכול לחוק בה עגול שיהיה מושש לכל צלעיה | |
|
ואם אין צלעיה וזוויותיה שוות יש מהם שלא יתכן לחוק בעגול שיהיה מושש לכל צלעיה | |
|
ואם תבא לידך צורה מכל התמונות מן משולש או מרובע או מחומש או למעלה מהם ואתה מחיק בתוכה עגולה מוששת לצלעיה הוי יודע כי רבוע חצי קוטר העגול ההוא במחצית כל צלעי התמונה הוא תשברתה | |
|
ואין אנו צריכין לתת דמיון מן המשולש ולא מן המרובע כי כבר פורש דרך רבועם | |
|
אבל אנו נותנין דמיון מן המחומש והוא אשר על חמש זוויותיו א'ב'ג'ד'ה' וכל צלע וצלע מצלעותיו שוות באורכן וכן זוויותיו כלם שוות זו לזו | |
|
ואנו מעגילים בתוכן עגול מושש לה' צלעותיו על חמש נקודות ז'ח'ט'כ'ל' | |
|
וציר העגול הזה הנקרא מרכז הוא נקודת מ' | |
|
ואנו אומרים כי רבוע חצי קוטר העגול הזה במחצית כל צלעי המחומש הוא תשברתו | |
|
וחצי הקוטר הוא קו יוצא מנקודת מ' אל אחת הנקודות אשר העגול מושש עליהם את המחומש | |
|
ועתה אם אנו מוצאים קו מנקודת מ' אשר הוא המרכז על שתי נקודות א' וב' מן זווית המחומש נתן לנו משולש אשר עליו מ'א'ב' | |
|
ותשבורת המשולש הזה הוא ברבוע העמוד היוצא אל תושבתו בחצי התושבת | |
|
ואם אנו מוציאים קו מנקודת מ' אל נקודת ל' אשר משש עליה העגול את צלע א"ב יהיה הקו הזה עמוד במשולש מ'א'ב' והוא חצי קוטר העגול | |
|
ואם מרבע העמוד הזה בחצי צלע א"ב יהיה רבועו תשבורת המשולש | |
|
| ||
|
ועל הדרך הזה אתה יכול לעשות חמשה משולשים על [34]חמש צלעי המשולש המחומש ויהיה תשבורת כל משולש מהם ברבוע חצי הקוטר בחצי הצלע כאשר היה בצלע הזה ותשבורת חמש המשולשים כולם הוא תשבורת המחומש והוא מרבוע חצי הקוטר בחצי כל חמש הצלעות | |
|
והוא יהיה העניין לכל צורה כמוה אשר צלעותיה רבים או מעטים אם אתה מעגיל בתוכה עגול מושש לצלעיה יהיה תשברתה ברבוע חצי קוטר העגול במחצית הצלעות וזאת היא צורתה | |
|
ומפני שאין אתה יכול להוציא בכל תמונה ותמונה עגול שיהיה מושש לכל צלעיה אין הכלל הזה מספיק לך במדידת כל העודפות על ד' צלעות | |
|
ומפני זה אני נותן לך כלל אחר שיהיה נוהג לך בכל צורה אשר צלעותיה ישרות | |
|
הוי יודע כי כל תמונה שטוחה שצלעותיה ישרות מתחלקת למשולשים שהם חסרים מן מניין הצלעות שנים | |
|
כגון המרובע אשר לו ד' צלעות יתחלק לב' משולשים אשר מניינם פוחת ב' ממספר הצלעות | |
|
והמשושה יתחלק לד' משולשים | |
|
וכן למעלה מזה | |
|
ואתה בא מן הכלל הזה וחלק כל תמונה שיבוא לידך אל המשולשים אשר היא נחלקת אליהם ורבע כל משולש מהם על הדרך אשר למדת ויהיה מרובע כולם תשבורת התמונה אשר נחלקו ממנה | |
|
כאלו היית מוצא במחומש אשר ציירתי לך למעלה קו מנקודת א' אל נקודת ג' וקו אחר אל נקודת ד' ויהיה המחומש נחלק לג' משולשים | |
|
ואין אתה יכול לפחות מהמשולשים האלה | |
|
ואם אתה מרבע אותם יהיה רבוע שלשתם תשבורת המחומש כגון הצורה הזאת | |
Square |
||
|
קלא הרי שיש לפניך א' מרובע מי' אמה על י' אמה ובאמצע הוא חקוק ופוחת ד' אמות על ד' אמות ותרצה לדעת כמה הוא המרובע בשיבור אחר הוצאת הד' אמות על ד' אמות מן החקק | |
|
|
||
|
תאמר אלו היה הטבלה מרובעת שלימה בלתי שום חקיקה היית אומר י' פעמ' י' הם ק' ותשברתו היה ק' | |
|
ועתה שפוחת באמצע ד' על ד' תאמ' ד' פעמ' ד' הם י"ו | |
|
תפחתם מק' ישארו פ"ד | |
|
| ||
|
א"כ א' טבלה מרובעת שהיא י' על י' ופוחת באמצעה ד' על ד' תשברתה פ"ד | |
|
וכן כל הדומה לו | |
Scalene Trapezoid | ||
|
[35]קלב קטומה שיש לה ב' קוים נכוחיים קו א"ג ואורכו ח' אמות וקו ב"ז ואורכו כ"ב אמות וב' צלעים שאינם שוות והם קו א"ב ואורכו ט"ו וקו ג"ד ואורכו י"ג אמות והצורה הזאת נקראת קטומת הראש חלופה | |
|
וריבוע הצורה הזאת יהיה בהוצאת עמוד ה' כאשר עשית בראשונה שכתבתיה למעלה | |
|
ואתה צריך בקטומה הזאת לגבל מעמד העמודים ולדעת מעמד הגדול ומעמד הקטון ואתה נוהג בהם המנהג הזה | |
|
תרבע הצלע הקצורה וגרע מרובעה ממרובע הצלע הארוך והעודף שבהם תקח מחציתו ותחלק אותו על עודף התושבת על הראש ואשר יצא מהחלוקה הזאת אם אתה מוסיף אותו על מחצית עדף התושבת תמצא המעמד הגדול אשר לעמוד והוא מרחקו מן הצלע הארוך | |
|
| ||
|
ואם תגרעינו ממחצית העודף תמצא המעמד הקטן אשר לעמוד והוא מרחקו מן הצלע הקצר | |
|
| ||
|
ואם אתה מרבע בצורה הזאת י"ג שהוא אורך צלע ג"ד הקצור יהיה מרובעו ק'ס'ט' | |
|
פחות אותו ממרובע א"ב שהוא מרובע הצלע הארוך ישאר נ"ו | |
|
קח מחצית המניין הזה והוא כ"ח | |
|
חלק אותו על י"ד שהוא עודף התושבת על הראש תהיה החלוקה ב' | |
|
ואם תוסיף ב' על ז' אשר הוא חצי עדף התושבת יהיה ט' והוא אורך קו ב"ה אשר הוא מרחק העמוד מהצלע הארוך | |
|
| ||
|
ואם תפחות ב' מז' תמצא מרחק העמוד מהצלע הקצר | |
|
והאות על העניין הזה התבונן לך מאשר פירשתי למעלה בעמוד המשולש מתחלף הצלעות | |
|
|
||
|
כי בהוציאך מן התושבת את הרחב הראש והוא ה' ישאר קו ט"ח ג' אמות פחות שביע והוא עודף מעמד עמוד המשולש על מעמד עמוד הקטומה הקצר | |
|
והעודף הזה רבע בצלע הקטומה הקצר שהוא י"ג יהיה ל"ז ושביעית | |
|
בוא וחלק המספר הזה על מעמד העמוד הקצר בקטומה והוא ה' תהיה החלוקה הזאת ז' וג' שביעיות והוא עודף צלע המשולש על צלע הקטומה הקצרה והוא קו ג"ז היוצא מצד הקצר | |
|
| ||
|
ואם תרצה לדעת קו א"ז הארוך הוצא המעמד הארוך במשולש על העניין שהוצאת הקצר | |
|
ותמצא אותו י"ד ושביעית והוא מעדיף על מעמד הארוך ה' ושביעית | |
|
מנה אותו בצלע הארוך שהוא ט"ו יהיה המניין ע"ז ושביעית | |
|
חלק המספר הזה על ט' שהוא המעמד הארוך בקטומה תהיה החלוקה ח' וד' שביעיות והוא עודף צלע המשולש על צלע הקטומה הא' הארוך והוא א"ז | |
|
| ||
|
ואם תבוא לדעת עמוד המשולש הזה הוי מונה עודף המעמד הקטן והוא ג' פחות שביע בעמוד הקטומה שהוא י"ב | |
|
וחלק אותו על מעמד הקטן והוא ה' | |
|
או הוי מונה עודף המעמד הגדול והוא ה' ושביע בעמוד הקטומה שהוא י"ב | |
|
נחלקהו על המעמד הגדול והוא ט' | |
|
תמצא שניהם מוציאים אל חשבון אחד והוא ז' פחות שביע והוא עודף עמוד המשולש על עמוד הקטומה | |
|
הוסף אותו על י"ב שהוא עמוד הקטומה ויהיה הכל י"ט פחות שביע והוא אורך קו ז"ט אשר הוא עמוד המשולש בצורה הזאת | |
|
| ||
|
[36]גליון וזה דבר ברור הוא כאשר אמרנו למעלה | |
|
כי קו ה"ט בקו א"ה כקו ב"ה בקו עודף עמוד המשולש על עמוד הקטומה שהיה א"ה | |
|
על כן אם נרבע קו ה"ט בקו א"ה ונחלק על קו ב"ה יצא לנו העודף | |
Right Trapezoid |
||
|
קלג התמונה השלישית הקטומה שראשה נכוחי לתושבתה ואחד מצלעיה עמוד עליה והתמונה הזאת נקראת חצי קטומה והדמיון לצורה הזאת קטומה שעליה א'ב'ג'ד' וראשה א"ג והיא ח' ותושבתה ב"ד והיא כ' וצלע א"ב הארוך ט"ו וצלע ג"ד הקצור ט' אמות והוא עמוד על תושבת ב"ד | |
|
וחשבון רבוע הקטומה הזאת הוא באסיפת ראשה אל תושבתה ולקחת מחציתם וימנה בעמוד ויהיה תשבורת הקטומה | |
|
ואם תאסוף בצורה הזאת ראשה אל תושבתה יהיה כללם כ"ח ומחציתם י"ד | |
|
ואם תרבע המספר הזה באורך העמוד והוא ט' יהיה ק'כ'ו' והוא תשבורת הקטומה הזאת | |
|
ואות לזה מפורש למעלה בקטומה הנשלמת | |
|
ואם תבוא לדעת אלכסונה הקצור והוא א"ד | |
|
הוי מרבע את הראש והוא ח' ומרובעו ס"ד | |
|
ואסוף אליו מרובע העמוד והוא הצלע הקצור והוא פ"א ויהיו שניהם יחד ק'מ'ה' והוא מרובע האלכסון | |
|
וגדר המספר הזה הוא אורך אלכסון הקצור | |
|
| ||
|
ואם תרצה לדעת אלכסון הארוך | |
|
אסוף מרובע התושבת למרובע העמוד ויהיו שניהם ת'פ'א' והוא מרובע אלכסון הארוך | |
|
| ||
|
ונתכוון לך החשבון הזה מפני ששתי זוויות אשר על נקודת ג' ונקודת ד' מן צלע ג"ד הן ניצבות כי הצלע הזה הוא עמוד על התושבת ועל הראש אשר הם נכוחיים | |
|
ואלכסון א"ד הוא מיתר לזוית ג' הניצבה | |
|
ואלכסון ג"ב הוא מיתר לזוית ד' הניצבה | |
|
ומרובע מיתר זוית הניצבה הוא שוה לשם מרובעי ב' הצלעות אשר הן מקיפות לה כאשר ידעת | |
|
וזה לך האות על חשבון האלכסונות בצורה הזאת | |
|
ואם תרצה להשלים הקטומה הזאת ולהוציא ראש המשולש הנקטמת ממנה | |
|
עשה כמעשה שלמדת מן הקטומה שלפניה | |
|
והוא שתהיה יודע עודף התושבת על הראש והוא י"ב בצורה הזאת | |
|
ואם תמנה הראש בקו א"ב וחלקנו על עודף התושבת יצא לך אורך הקו היוצא מהעמוד אל ראש המשולש | |
|
ואתה יכול להוציאו למעשה מן הדרך שלמדת בצורות האחרות | |
Obtuse Trapezoid |
||
|
קלד קטומה שראשה נכוחי לתושבתה והצלע אחת נופלת על התושבת על זוית נרווחת ולקטומה הזאת שני עמודים תכונים ועמוד חצוני | |
|
ואתה יודע תשבורת הקטומה הזאת בהוצאת עמודיה | |
|
ואתה צריך תחלה להגביל מעמד העמוד | |
|
והוא שתהיה פוחת הראש והוא י"ד מן התושבת והוא כ"א ישאר בידך ז' והוא עודף התושבת | |
|
ובוא ורבע העודף הזה ואסוף מרובעו אל מרובע הצלע הקצור ותמצא ב' מרובעים אילו ר'ע'ד' | |
|
הוצא המספר ממרובע הצלע הארוך שהוא ת' ישאר בידך ק'כ'ו' | |
|
קח המחצית [37]והוא ס"ג חלק אותו על ז' והוא עודף התושבת | |
|
ויהיה הכל י"ו והוא גבול מעמד העמוד מנגד קו א"ב הארוך | |
|
ואלו הט' אשר מצאת הם המרחק הקצר | |
|
| ||
|
וכן מרחק העמוד שעליו ג"ח אשר הוא יוצא חוצה מן קו ב"ד התושבת וכן מרחק העמוד התיכוני הנופל על נקודת ד' שעליו ד"ט | |
|
ואם תבוא לדעת אורך העמוד | |
|
הוי מרבע את מעמד שתרצה | |
|
ואם [אתה] מרבע המעמד הארוך שהוא י"ו הוציאנו ממרובע הצלע הארוך אשר הוא כ' | |
|
ואם תרבע המעמד הקצור הוציאנו ממרובע הצלע הקצר | |
|
ויהיה המותר מן כל אחד משני החשבונים האלה ק'מ'ד' והוא מרובע העמוד בק | |
|
וגדרו י"ב והוא אורך העמוד | |
|
| ||
|
ותשבורת הקטומה הוא ברבוע העמוד בחצי הראש ובחצי התושבת כאשר עשית בשאר הקטומות שלפניה | |
|
והתשבורת הוא ר"י | |
|
והאות על החשבון הזה שהוצאת בו את העמוד הוא מעניין האות שהורתיך בקטומה שאין צלעותיה שוות | |
|
כי בהוציאך הראש מהתושבת ונשארו בידך ז' היה הקו ההוא צלע למשולש מרויח הזויות א' שצלעו האחת ז' והשינית ט"ו והם הצלעות המקיפות זוית הנרווחת | |
|
ומיתר הזוית הזאת הוא כ' אמה אשר הוא אורך קו א"ב מן הקטומה הזאת | |
|
ואם אתה מוציא במשולש הזה המרויח הזויות עמוד שיהיה נופל חוצה אתה מוצא מעמדו חוצה כאשר מצאת בחשבונך בצורה הזאת | |
|
|
||
|
ואתה יכול להוציא אלכסון הקטומה הארוך אם אתה מאסף אל התושבת מעמד העמוד החיצוני וכולל את הכל ומרבע אותו | |
|
כאילו היית מאסף בצורה הזאת כ"א שהוא אורך התושבת אל ט' שהוא אורך מעמד העמוד חוצה ויהיה הכל ל' | |
|
ואתה מרבע המניין הזה ומוסיף עליו מרובע העמוד ויהיה הכל אלף ומ"ד והוא מרובע האלכסון הארוך | |
|
וגדר המספר הזה הוא אורך האלכסון בקטומה הזאת מנקודת ג' אל נקודת ב' | |
|
| ||
|
ואם תרצה להוציא אלכסון הקצור היוצא מן א' ועד ד' | |
|
תאסוף אל מרובע העמוד מרובע עודף התושבת על המעמד הארוך והוא בצורה הזאת ה' ויהיו שני המרובעים ק'ס'ט' והוא מרובע האלכסון הקצור | |
|
וגדרו הוא אורך האלכסון | |
|
| ||
|
והאות אל העניין הזה אתה יכול להבין מהאותות אשר למעלה אם אתה מביט אל המשולשים מוצבי זוית אשר הם מתיילדים מהאלכסונות האלה עם צלעי הקטומה והעמודים כאשר הראיתך למעלה | |
|
ויכול הוא בצורה הזאת שיהיה אלכסון הקצור הוא עמוד בקטומה בעצמו | |
|
כאילו היה בצורה הזאת אורך ראש הקטומה ט' אמות ותושבתה י"ו ומניין ב' הצלעות הנשארות המניין אשר היה להם בצורה הראשונה | |
|
והיית מוצא בצורה הזאת השינית העמוד נופל מנקודת א' אל נקודת ד' ויהיה העמוד ההוא אורך האלכסון הקצר | |
|
והאלכסון הארוך אשר הוא יוצא מג' עד ב' הוא גדר ת'ש'ס'ט' אשר הוא מרבע עמוד א"ד [38]עם מרובע קו א"ג עם קו ב"ד אשר ראש הקטומה ותושבת שניהם יחד בקו אחד | |
|
| ||
|
ואתה מבין אותות כל החשבון הזה על מכונו אם אתה מעיין בה עיון יפה | |
Quadrangle which has no parallel sides |
||
|
קלה המין השני הנשאר מן התמונות אשר יש להם ד' צלעות הוא כל מרובע שאין אחת מצלעותיו נכוחי לצלע אחרת | |
|
ובתמונות האלו אי אתה מוצא רבועם אלא מרבוע המשולשי' אשר הם נחלקי' עליהם | |
|
וכל מרובע בעולם אתה יכול לחלקו לשני משולשין | |
|
וידוע הוא כי המשבר שני המשולשי' ההם ומצרף תשברתם ימצא התשבורת המרובע אשר הם חלקיו | |
|
ועל הענין הזה תוכל למצא התשבורת כל מרובע על חלופי מיניהן מן השוים בצלעותיו והנכוחיים מתשבורת המשולשים שהן נחלקים עליהם | |
|
אלא שהמרובעים השוים בצלעותיו והנכוחיים בהם אתה יכול להגיע אל תשברתן מדרך אחרת ואינך צריך לרבע בהן את המשולשים הנחלקים עליהם | |
|
ושאר המרובעים הנפתלים ואין בהם צלע נכוחי ובצלע אחד אי אתה יכול למצוא תשברתם אלא מתשבורת המשולש אשר יחלקו עליהם | |
|
ואני נותן לך דמיון בעניין זה ויהיה מספיק לך בשאר הצורות והדמיון הזה הוא מהצורה שהשלמתי פירושה | |
|
| ||
|
והיא הקטומה הרביעית המתמוטטת אשר צלע א"ג היה בה י"ד אמה וצלע ב"ד כ"א אמה וצלע א"ב כ' אמות וצלע ג"ד ט"ו אמה | |
|
ומצאת האלכסון הקצור בקטומה הזאת כאשר חשבונו למעלה י"ג אמה | |
|
וכשמדדת אותו אתה מרבע לא מצאת בו המידה הזאת אלא יותר ממנה או פחות ממנה | |
|
ואנו מוסרין כאילו מצאת אותה יתר ב"ג י"ו אמה ונודע לך מזה כי אין הצורה הזאת קטומת הראש כי אין אחת מצלעיה נכוחית עם צלע אחרת לפי אם היו נרוחיות לא היה אלכסונה הארוך או הקצר מתחלף מחשבונו שהיא בקטומה | |
|
|
||
|
ומפני זה אתה צריך לרבע שני המשולשים אשר נחלק עליהן המרבע הזה באלכסון שהוצאת בו והוא שני משולשי א'ד'ב' וא'ד'ג' וכל אחד מהם צלעותיו ידועות | |
|
ואתה יכול להגיע אל ריבועם בהוצאת העמודים כאשר למדת בריבוע המשולשים | |
|
ואם אתה נוהג בהם המנהג הזה תמצא תשבורת משולש א'ד'ב' מהן צ"ו ושליש פחות משהו | |
|
ומשולש א'ד'ג' תהיה תשברתו ק"נ וב' שלישיים פחות משהו | |
|
ויהיה כל התשבורת בעניין הזה ר'מ'ז' פחות משהו | |
|
ואנו מצאנו רבועו בקטומה המתמוטטת ה' ונמצא הריבוע עודף בתמונה הזאת מפני עדיפת האלכסון | |
|
ואילו היה האלכסון מתמעט היה הריבוע פוחת | |
|
ומהעניין הזה אתה יכול למסור לך גדול במרובעת | |
|
ואומר מרובע אשר אתה מוציא את אלכסונו נחלק לב' משולשים | |
|
אם אתה מוציא עמוד של האלכסון בשני המשולשין ותאסוף את שני העמודים ותקח את מחציתם ותצרף המחצית הזה במניין כל האלכסון אתה מוציא תשבורת המרבע | |
|
או תצרף שני העמודים במחצית האלכסון וגם בא תמצא תשבורת המרובע | |
|
ואיני צריך להביא לך [39]להביא לך משל מן החשבון הזה מפני שאתה מבין אותו מהדמיונות אשר במשיחת המשולשין ואיני נזקק להאריך בפירוש המרובעי' יותר מזה | |
Additional Problems |
|
| Motion Problem - Pursuit | |
|---|---|
|
קלו אם יאמר הרי אדם שהוא מהלך י' מלים בכל יום ואחר הוא מהלך ביום ראשון א' מיל וביום שני ב' מילים וביום השלישי ג' ומוסיף והולך בכל יום |
|
|
|
תכפול המילים שמהלך הקבוע יהיו כ' |
|
וגרע מהם אחד ישארו י"ט הרי שבי"ט ימים יהיו בשוה |
|
וכן לעולם תכפול שמהלך הקבוע ותגרע מהם אחד |
|
קלז ואם יאמר הרי אדם שהוא מהלך י' מילים בכל יום והאחר מהלך ביום אחד א' מיל וביום שני ג' וביום שלישי ה' וביום רבעי ז' ומוסיף והולך בכל יום חשבון נפרד |
|
דע כי כמספר המילים שמהלך הקבוע ליום כן מספר הימים שיעמדו בשוה |
|
ואם הקבוע מהלך י' מילים ביום בי' ימים יעמדו בשוה |
|
ואם מהלך ט' מילים ביום בט' ימים יעמדו בשוה וכה"ל |
|
קלח וכן אדם שמהלך בכל יום י' מילים והאחר מהלך ביום ראשון ב' וביום שני ד' וביום שלשי ו' ומוסיף והולך בכל יום מספר זוג כגון בדוח"י י"ב י"ד י"ו |
|
תחסר אחד מן המילים שמהלך הקבוע שהם י' יהיו ט' הרי שבט' ימים יעמדו בשוה |
|
וכן לעולם תחסר אחד ממספר המילים וכן כל הדומה לו |
| Triangulation Problem - Tree | |
|
שאלה אילן שגובהו י' אמות ובא רוח חזק ושברתו לשנים חלקים ולא נערכו חלקיו אך נטז ממקו' השבירה והגיע ראשו לארץ |
|
תשובה תחלק מרובע הרוחק שמשרש האילן לראש שהם כ"ה במשלנו על גובה האילן שהם י' ויצאו ב' וחצי |
|
נחברם עם הי' ונקח חציים שהם ו' ורביע וזהו כמות האילן עד מקום השבירה |
|
| |
|
והנשאר עד תשלום הי' שהם ג' וג' רביעיות הם כמות האמות שממקום השבירה עד שרש האילן |
Additional Problems - MS Mantova |
|
| Multiple Quantities - Boys Sharing Property of Their Father | |
|
[40]אדם שהיו לו בנים ולא נודע מספרם ואמר לאחד לך בארגז וקח לך פרח אחד והעשירית מכל הנשאר |
|
| |
|
לפי חשבון השבור [.....] הוא עשירית |
|
תפחות א' מעשרה ישארו ט' הרי שט' היו הבנים |
|
אמור אחר זה ט' פעמי' ט' הם פ"א וככה היו הפרחים |
|
ואם יקחו כאשר צוה אביהם יהיה לכל אחד חלק שוה |
|
וכן לכל חשבון שתרצה עשה כן שתפחות א' מהחשבון השבור והוא חשבון הבנים ותרבע אותו והוא חשבון הפרחי' וכמספר הבנים יהיו [41]מספר הפרחים שיבא לכל אחד ודו"ק |
| MS Mantova; MS Amsterdam: Multiple Quantities - Boys Selling Cubits of a Cloth | |
|
הרי אדם שנתן לג' בניו ל' אמות בגד והלכו לשוק למכרו האחד מכר בד' דנרי' האמה והשני בה' והשלישי בו' |
|
אומר תחילה רביע וחומש ושתות נמצא בס' |
|
וכללם הוא ל"ז והוא המחלק |
|
ואם תרצה לדעת כמה אמות מכר אותו שמכר בד' דינרי' |
|
תפוס הרביע מס' שהוא ט"ו ותרבע אותו על סך הבגד שהוא ל' ואמור ט"ו פעמי' ל' ת"נ |
|
חלקם בל"ז יבא י"ב ו' חלקי' מל"ז |
|
הרי שמכר [הה] י"ב אמות ו' חלקי' מל"ז |
|
ואם תרצה לדעת כמה מעות קבל |
|
אמור תחילה מי"ב אמות קבל מ"ח דינ' |
|
ומן הו' חלקי' מל"ז עשה על זה הדרך אם ל"ז שהוא אמה שלמה שוה מ"ח פשי' הו' חלקי' מל"ז כמה שוים |
|
אמור ו' פעמי' מ"ח רפ"ח |
|
חלקם בל"ז יבא ז' פשי' וכ"ט חלקים מל"ז |
|
וכזה תעשה האחרי' ויצא לך העניין |
Apparatus
Appendix: Bibliography
Anonymous
Sefer ha-Kelalim ba-Mispar
Manuscripts:
- 1) Amsterdam, Portugees Israelitisch Seminarium Ets Haim 47 D 20/43 (IMHM: f 3576), ff. 227r-232v (15th century)
- Ets Haim 47 D 20/43
- 2) Cambridge, University Library Add. 553 (IMHM: f 16842), ff. 90r-95r; 101r-104r (17th century)
- 3) Mantova, Comunità Ebraica MS ebr. 8/16 (IMHM: f 788), ff. 72r-82v (Porto, 1464-1471)
- ebr. 8/16
- 4) Moscow, Russian State Library, Ms. Guenzburg 698/2 (IMHM: f 47914), ff. 10r-40v (14th-15th century)
- 5) Oxford, Bodleian Library MS Mich. 536/6 (IMHM: f 22082), ff. 88r-99v; 110r-117v (cat. Neub. 1268, 6); (1518-1520)
The transcript is based mainly on manuscript Oxford 536
Bibliography:
Steinschneider, Moritz. 1906. Mathematik bei den Juden, Band II: 1551-1840. Monatsschrift für die Geschichte und Wissenschaft des Judenthums 50, p. 214. repr.: ed. Gad Freudenthal, Hildesheim, Zürich, New York: Olms, 2014, p. 137.
![\scriptstyle{\color{OliveGreen}{\frac{1}{a}-\frac{1}{b}=\frac{\left[\frac{1}{a}\sdot\left(a\sdot b\right)\right]-\left[\frac{1}{b}\sdot\left(a\sdot b\right)\right]}{a\sdot b}}}](/mediawiki/images/math/c/0/6/c0629aecee310d90e051b57b2c593d46.png)
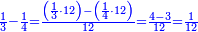
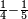
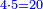

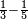
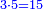
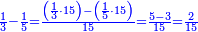
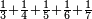
![\scriptstyle{\color{blue}{\left[\left[\left(3\sdot4\right)\sdot5\right]\sdot6\right]\sdot7=\left[\left(12\sdot5\right)\sdot6\right]\sdot7=\left(60\sdot6\right)\sdot7=360\sdot7=2520}}](/mediawiki/images/math/6/0/8/6087f1e952aeacad37a6f010aec8851d.png)
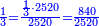
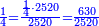
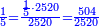
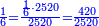
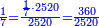

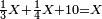
![\scriptstyle{\color{blue}{12-\left[\left(\frac{1}{3}\sdot12\right)+\left(\frac{1}{4}\sdot12\right)\right]=12-7=5}}](/mediawiki/images/math/9/9/4/994410b776b40d19b554ba92d759d4ae.png)
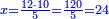
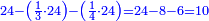
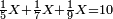
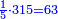
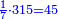
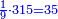
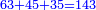
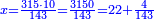
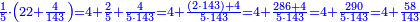
![\scriptstyle{\color{blue}{\left[\frac{1}{5}\sdot\left(22+\frac{4}{143}\right)\right]+\left[\frac{1}{7}\sdot\left(22+\frac{4}{143}\right)\right]+\left[\frac{1}{9}\sdot\left(22+\frac{4}{143}\right)\right]=10}}](/mediawiki/images/math/3/6/5/3657322cf92ee517a021923fd6effa87.png)
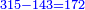
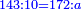
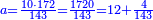
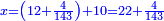
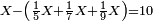
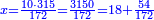
![\scriptstyle{\color{blue}{\left(18+\frac{54}{172}\right)-\left[\left[\frac{1}{5}\sdot\left(18+\frac{54}{172}\right)\right]+\left[\frac{1}{7}\sdot\left(18+\frac{54}{172}\right)\right]+\left[\frac{1}{9}\sdot\left(18+\frac{54}{172}\right)\right]\right]=10}}](/mediawiki/images/math/1/0/f/10f16522c9ec6736af054beb8758e910.png)
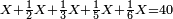
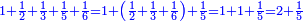

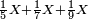
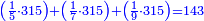
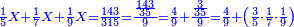
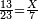
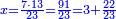
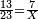
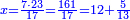
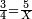
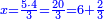 of Cortona
of Cortona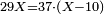
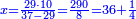
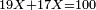
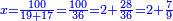
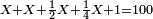
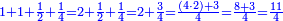
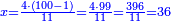
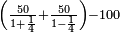
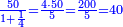
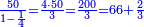
![\scriptstyle{\color{blue}{\left(\frac{50}{1+\frac{1}{4}}+\frac{50}{1-\frac{1}{4}}\right)-100=\left[40+\left(66+\frac{2}{3}\right)\right]-100=6+\frac{2}{3}}}](/mediawiki/images/math/5/4/e/54e344679b45d90141508c4ca499d0a6.png)


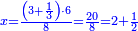
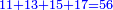
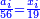


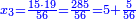
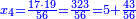
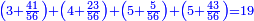
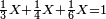
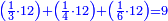
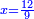
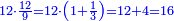
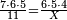
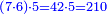
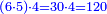
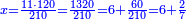
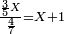
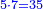
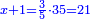
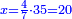
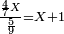

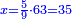
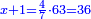

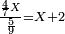


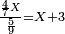


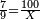
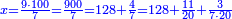
![\scriptstyle{\color{OliveGreen}{\frac{a_1}{b_1}:\frac{a_2}{b_2}=a_3:x\longrightarrow\left[\frac{a_1}{b_1}\sdot\left(b_1\sdot b_2\right)\right]:\left[\frac{a_2}{b_2}\sdot\left(b_1\sdot b_2\right)\right]=a_3:x\longrightarrow x=\frac{\left[\frac{a_2}{b_2}\sdot\left(b_1\sdot b_2\right)\right]\sdot a_3}{\frac{a_1}{b_1}\sdot\left(b_1\sdot b_2\right)}}}](/mediawiki/images/math/9/2/0/920461717fa923d2d19528270f52a2f0.png)
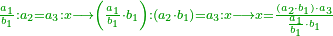
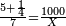
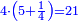
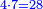

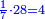
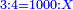
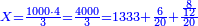
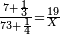
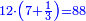
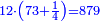
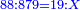
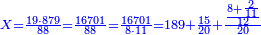



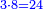

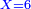
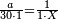
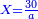

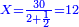
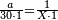
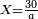
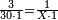
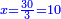
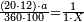
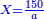
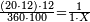
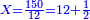

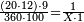

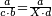
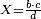
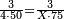
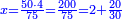
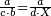
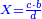
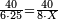
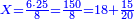
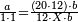
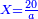
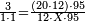
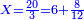
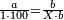
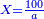
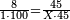
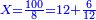
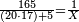
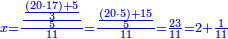

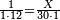
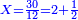
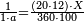
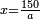
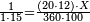
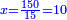

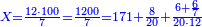
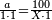
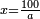
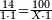

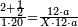
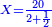
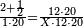
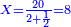
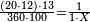
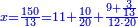
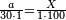
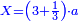
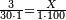
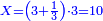
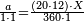


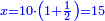

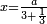
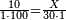
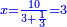

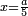


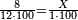
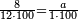
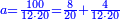
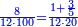
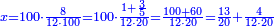
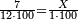
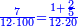
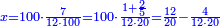

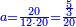

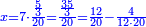
![\scriptstyle20+4-X+\left[\frac{4}{20}\sdot\left(20+4-X\right)\right]-X+\left[\frac{4}{20}\sdot\left[20+4-X+\left[\frac{4}{20}\sdot\left(20+4-X\right)\right]-X\right]\right]=X](/mediawiki/images/math/6/0/5/6053a7f78280380c9d2df6cfc64c3c4f.png)

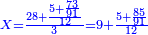
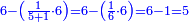
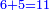
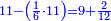
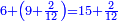
![\scriptstyle{\color{blue}{\left(15+\frac{2}{12}\right)-\left[\frac{1}{6}\sdot\left(15+\frac{2}{12}\right)\right]=12+\frac{7+\frac{2}{3}}{12}}}](/mediawiki/images/math/e/0/4/e04d582e4f2552e03545f8aea59a9acc.png)
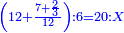
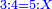
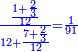
![\scriptstyle{\color{blue}{\left(12+\frac{7+\frac{2}{3}}{12}\right)-\left[\frac{1}{91}\sdot\left(12+\frac{7+\frac{2}{3}}{12}\right)\right]=12+\frac{1}{2}}}](/mediawiki/images/math/0/0/1/001e79fcd8b418bff28beebf4357d454.png)
![\scriptstyle{\color{blue}{\left(12+\frac{1}{2}\right)-\left[\frac{1}{5}\sdot\left(12+\frac{1}{2}\right)\right]=10}}](/mediawiki/images/math/5/1/3/513a14bb897c7a135c180395427a107b.png)

![\scriptstyle{\color{blue}{2\sdot\left[\left[6-\left(\frac{1}{91}\sdot6\right)\right]-\left[\frac{1}{5}\sdot\left[6-\left(\frac{1}{91}\sdot6\right)\right]\right]\right]=9+\frac{5+\frac{85}{91}}{12}}}](/mediawiki/images/math/a/f/2/af2f9548897783cb78fe90e3cbf580ef.png)
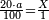
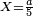
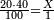
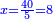


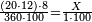
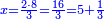
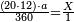
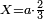
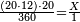
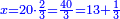
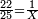
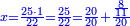
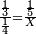
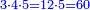



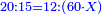

![\scriptstyle\left(\frac{1}{2}X+\frac{1}{3}X\right)+\left[\left(\frac{1}{3}+\frac{1}{4}\right)\sdot\left[X-\left(\frac{1}{2}X+\frac{1}{3}X\right)\right]\right]+3=X](/mediawiki/images/math/d/2/7/d276452e72993e94f14cd2ca6943159c.png)
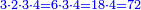
![\scriptstyle{\color{blue}{72-\left[\left(\frac{1}{2}\sdot72\right)+\left(\frac{1}{3}\sdot72\right)\right]=72-60=12}}](/mediawiki/images/math/0/d/c/0dc83d6b1571da9c902b55fe76608387.png)

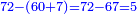

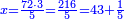
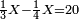


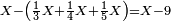
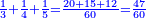
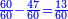
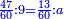
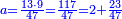
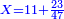
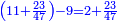
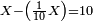
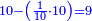

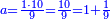

![\scriptstyle{\color{blue}{11+\frac{1}{9}-\left[\frac{1}{10}\sdot\left(11+\frac{1}{9}\right)\right]=10}}](/mediawiki/images/math/3/9/7/3977bd1a0169a0611a83ac0edf208b44.png)
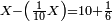
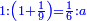
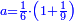

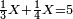
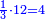

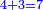
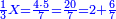
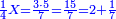
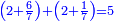

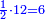

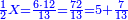
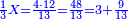
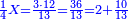
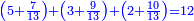

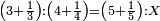
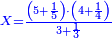
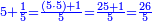

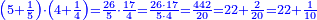

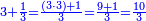
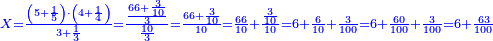
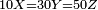
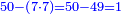

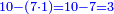

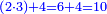

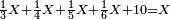
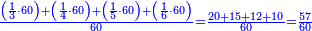
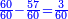
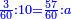
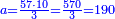

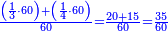
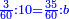
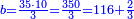
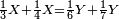
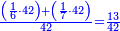
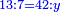
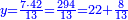
![\scriptstyle{\color{blue}{\left[\frac{1}{6}\sdot\left(22+\frac{8}{13}\right)\right]+\left[\frac{1}{7}\sdot\left(22+\frac{8}{13}\right)\right]=7}}](/mediawiki/images/math/7/b/8/7b872fc19ecb7a6fb8b3ed6bc94a0c38.png)
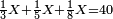








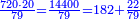
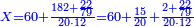
![\scriptstyle2\sdot\left[2\sdot\left[2\sdot\left(2x-8\right)-8\right]-8\right]=8](/mediawiki/images/math/b/7/c/b7c0d41d3d5b00c11ff0cfe8764fe1d2.png)
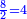

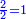

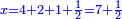
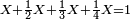

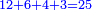

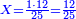
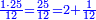
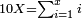
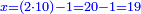
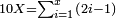
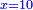
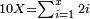
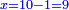
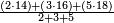
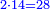


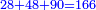
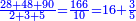
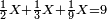
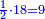
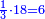
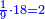


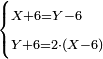


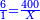

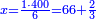
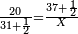
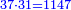
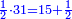
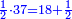
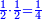
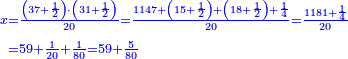

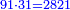
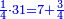
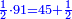

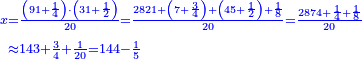

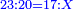

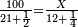

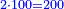
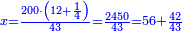
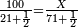
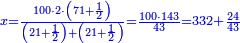
![\scriptstyle\left[\left(2-\frac{5}{3}\right)\sdot X\right]+\left[\left(3-\frac{5}{3}\right)\sdot X\right]=5](/mediawiki/images/math/b/3/9/b39a89f5f9f01ae578ff87165913ffb8.png)

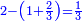
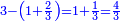

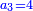
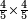
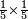
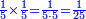
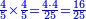
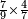
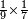
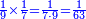
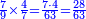
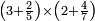

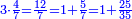
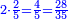
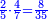
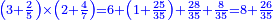

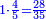
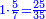
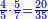
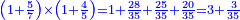
![\scriptstyle\begin{cases}\scriptstyle a_1+a_2+a_3+a_4=80\\\scriptstyle a_1=\left(\frac{1}{2}+\frac{1}{6}\right)\sdot a_2\\\scriptstyle a_2-\left[\left(\frac{1}{2}+\frac{1}{6}\right)\sdot a_2\right]=\frac{2}{3}a_3\\\scriptstyle a_3=\left(\frac{1}{3}+\frac{1}{4}+\frac{1}{6}\right)\sdot a_1\\\scriptstyle a_4=\frac{4}{5}a_3\end{cases}](/mediawiki/images/math/e/6/1/e61594e5bed663d7d2049121054974b5.png)
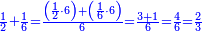
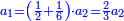



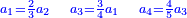
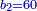


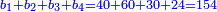

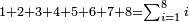
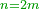 ]:
]:
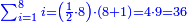

 ]:
]:![\scriptstyle{\color{OliveGreen}{\sum_{i=1}^n i=\left[\frac{1}{2}\sdot\left(n+1\right)\right]\sdot n}}](/mediawiki/images/math/f/0/5/f05c82d98b93f64fdea1180201df1e54.png)
![\scriptstyle{\color{blue}{\sum_{i=1}^7 i=\left[\frac{1}{2}\sdot\left(7+1\right)\right]\sdot7=4\sdot7=28}}](/mediawiki/images/math/a/7/c/a7cd0bfcbccb015720bd6dde810dd809.png)


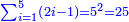
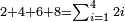
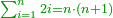
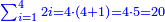
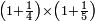

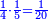

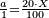

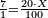

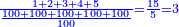
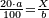

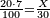
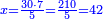
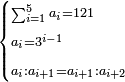
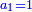
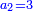
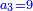



![\scriptstyle{\color{OliveGreen}{\left[\left(2\sdot1\right)+\left(4\sdot2\right)+\left(3\sdot4\right)\right]\bmod7=}}](/mediawiki/images/math/b/d/8/bd8cd8d9a5a69f4e75325dca7bdeca93.png) 1 → copper, gold, silver.
1 → copper, gold, silver.![\scriptstyle{\color{OliveGreen}{\left[\left(3\sdot1\right)+\left(2\sdot2\right)+\left(4\sdot4\right)\right]\bmod7=}}](/mediawiki/images/math/e/d/9/ed9c3072d779e894dc7d9bd6b1518106.png) 2 → silver, copper, gold.
2 → silver, copper, gold.![\scriptstyle{\color{OliveGreen}{\left[\left(2\sdot1\right)+\left(3\sdot2\right)+\left(4\sdot4\right)\right]\bmod7=}}](/mediawiki/images/math/d/2/9/d290492501dc1ca670dce7fdde74810a.png) 3 → copper, silver, gold.
3 → copper, silver, gold.![\scriptstyle{\color{OliveGreen}{\left[\left(4\sdot1\right)+\left(3\sdot2\right)+\left(2\sdot4\right)\right]\bmod7=}}](/mediawiki/images/math/d/b/8/db8690da87d43dafcdc91b8de3bc2800.png) 4 → gold, silver, copper.
4 → gold, silver, copper.![\scriptstyle{\color{OliveGreen}{\left[\left(3\sdot1\right)+\left(4\sdot2\right)+\left(2\sdot4\right)\right]\bmod7=}}](/mediawiki/images/math/1/7/a/17ae82029f9b50d7ab20ae9d9e3aed2c.png) 5 → silver, gold, copper.
5 → silver, gold, copper.![\scriptstyle{\color{OliveGreen}{\left[\left(4\sdot1\right)+\left(2\sdot2\right)+\left(3\sdot4\right)\right]\bmod7=}}](/mediawiki/images/math/4/0/f/40fea3c45e9afe81fc065fc0c9851fdf.png) 6 → gold, copper, silver.
6 → gold, copper, silver.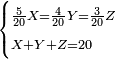
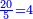
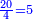
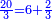
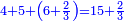 days
days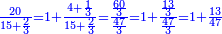

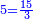
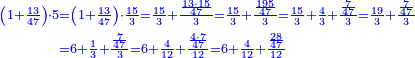
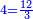
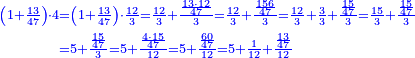
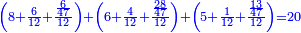
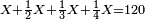
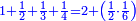
![\scriptstyle{\color{blue}{\left[2+\left(\frac{1}{2}\sdot\frac{1}{6}\right)\right]\sdot60=125}}](/mediawiki/images/math/f/e/e/feee1688775c37c50f8c73a0ca686bf5.png)
![\scriptstyle{\color{blue}{\left[2+\left(\frac{1}{2}\sdot\frac{1}{6}\right)\right]\sdot12=12+13}}](/mediawiki/images/math/c/8/4/c84d44d55e4f0aecc61dd6b809638c69.png)
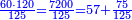
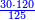
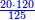
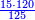

![\scriptstyle{\color{blue}{\left(\frac{1}{4}\sdot30\right)+\left[\frac{1}{3}\sdot\left(\frac{1}{4}\sdot40\right)\right]=\left(7+\frac{1}{2}\right)+\left(\frac{1}{3}\sdot10\right)=\left(7+\frac{3}{6}\right)+\left(3+\frac{2}{6}\right)=10+\frac{5}{6}}}](/mediawiki/images/math/8/0/2/8025548d7ffea180aaf74b2aa258a187.png)
![\scriptstyle{\color{blue}{\left(\frac{1}{4}\sdot30\right)+\left[\frac{1}{3}\sdot\left(\frac{1}{4}\sdot40\right)\right]+\left[\frac{1}{2}\sdot\left(\frac{1}{3}\sdot60\right)\right]=20+\frac{5}{6}}}](/mediawiki/images/math/6/3/9/639c2256db341185f9c23923c2b05f10.png)
![\scriptstyle{\color{blue}{\left(\frac{1}{4}\sdot30\right)+\left[\frac{1}{3}\sdot\left(\frac{1}{4}\sdot40\right)\right]+\left[\frac{1}{2}\sdot\left(\frac{1}{3}\sdot60\right)\right]+\left(\frac{1}{2}\sdot120\right)=80+\frac{5}{6}}}](/mediawiki/images/math/4/b/7/4b7c4c6a1a27b51b2f61f1452a8a65a0.png)
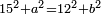
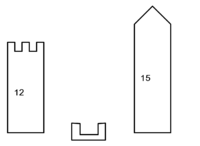

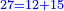
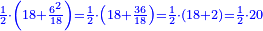

![\scriptstyle\scriptstyle{\color{OliveGreen}{\left[\frac{1}{2}\sdot\left(\rm{length_1+length_2}\right)\right]\sdot\left[\frac{1}{2}\sdot\left(\rm{breadth_1+breadth_2}\right)\right]}}](/mediawiki/images/math/0/5/4/0541cff5b82ef3ad6443caeeaba42f0c.png)
![\scriptstyle{\color{blue}{\left[\frac{1}{2}\sdot\left(4+6\right)\right]\sdot\left[\frac{1}{2}\sdot\left(5+3\right)\right]=\left(\frac{1}{2}\sdot10\right)\sdot\left(\frac{1}{2}\sdot8\right)=5\sdot4=20}}](/mediawiki/images/math/6/b/e/6be403d9d0da87e677138195512e3aaa.png)


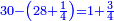


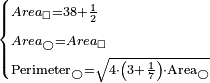
![\scriptstyle{\color{blue}{\sqrt{\left(38+\frac{1}{2}\right)\sdot\left[4\sdot\left(3+\frac{1}{7}\right)\right]}=\sqrt{\left(38+\frac{1}{2}\right)\sdot\left(12+\frac{4}{7}\right)}=\sqrt{484}=22}}](/mediawiki/images/math/7/b/7/7b7dca044063af8f374417378f76a6a1.png)
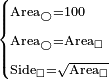

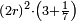
![\scriptstyle{\color{OliveGreen}{\rm{Surface_{sphere}}\sdot\left[\frac{1}{6}\sdot\left(2r\right)\right]}}](/mediawiki/images/math/1/c/5/1c52cc720d920cee8198165189e5281f.png)

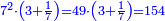
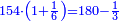
![\scriptstyle{\color{blue}{\left[7\sdot\left(3+\frac{1}{2}\right)\right]\sdot\left(3+\frac{1}{7}\right)=77}}](/mediawiki/images/math/7/4/5/7457b23543f85dd128179a780b2b2382.png)
![\scriptstyle{\color{blue}{77\sdot\left[\frac{1}{6}\sdot\left(2r\right)\right]=90-\frac{1}{6}}}](/mediawiki/images/math/4/d/2/4d2d28f827869eea6319d87dc150ff02.png)

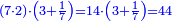
![\scriptstyle{\color{blue}{44\sdot\left[\frac{1}{6}\sdot\left(2r\right)\right]=44\sdot\left(1+\frac{1}{6}\right)=51+\frac{1}{3}}}](/mediawiki/images/math/4/c/c/4cc6a351ee80703dd6fdbd7965782c9e.png)
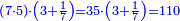
![\scriptstyle{\color{blue}{110\sdot\left[\frac{1}{6}\sdot\left(2r\right)\right]=128+\frac{1}{3}}}](/mediawiki/images/math/1/b/d/1bd0a32a24afbf4ad5cbbfff01b1a358.png)



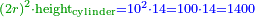
![\scriptstyle{\color{blue}{1400-\left[\left[\frac{1}{7}+\left(\frac{1}{2}\sdot\frac{1}{7}\right)\right]\sdot1400\right]=1400-300=1100}}](/mediawiki/images/math/9/6/8/968c893b6e831904d39545b4738b1cda.png)
![\scriptstyle{\color{blue}{100-\left[\left[\frac{1}{7}+\left(\frac{1}{2}\sdot\frac{1}{7}\right)\right]\sdot100\right]=100-\left(21+\frac{3}{7}\right)=78+\frac{4}{7}}}](/mediawiki/images/math/f/1/8/f18ddd8d864724be886adef0829db8d2.png)


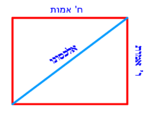
![\scriptstyle{\color{blue}{\sqrt{\left[2\sdot\left(8\sdot6\right)\right]+\left(8-6\right)^2}=\sqrt{\left(2\sdot48\right)+2^2}=\sqrt{96+2^2}=\sqrt{100}=10}}](/mediawiki/images/math/b/a/6/ba6094813180dc06f481c47295a2e833.png)

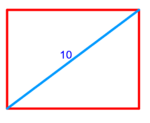
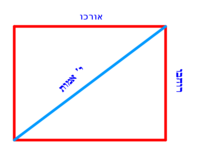
![\scriptstyle{\color{blue}{\frac{1}{2}\sdot\left[diagonal^2-\left(side_1-side_2\right)^2\right]=\frac{1}{2}\sdot\left(10^2-2^2\right)=\frac{1}{2}\sdot\left(100-4\right)=\frac{1}{2}\sdot96=48}}](/mediawiki/images/math/a/d/9/ad9a0fe0dcb662818d3ab0d7c95a9c00.png)



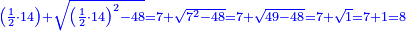
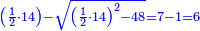
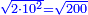
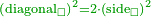
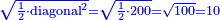

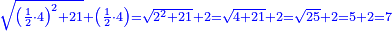

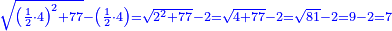
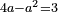
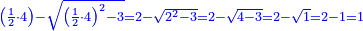
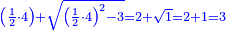
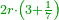
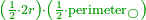

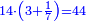
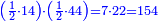

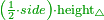

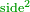
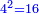

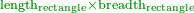
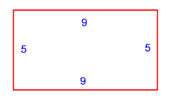
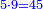
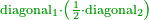
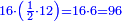
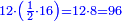
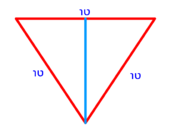
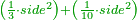
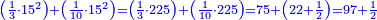

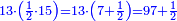
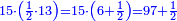

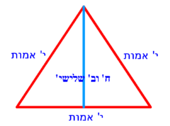
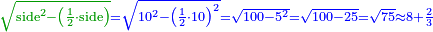
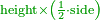
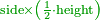
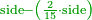

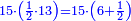
![\scriptstyle{\color{OliveGreen}{\sqrt{\rm{height}^2+\left[\frac{1}{3}\sdot\left(\rm{height}^2\right)\right]}}}](/mediawiki/images/math/1/b/8/1b868584da526e782eefe9f61ecdf63b.png)
![\scriptstyle{\color{blue}{\sqrt{13^2+\left[\frac{1}{3}\sdot\left(13^2\right)\right]}=\sqrt{169+\left(\frac{1}{3}\sdot169\right)}=\sqrt{169+\left(56+\frac{1}{3}\right)}=\sqrt{225+\frac{1}{3}}\approx15}}](/mediawiki/images/math/1/b/e/1be4eaaa35521be3c6f64424d6b16ded.png)
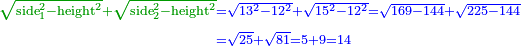

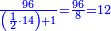

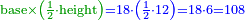
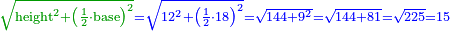
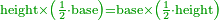
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle\frac{\frac{1}{2}\sdot\left[\left(AG^2+BG^2\right)-AB^2\right]}{BG}&\scriptstyle=\frac{\frac{1}{2}\sdot\left[\left(15^2+14^2\right)-13^2\right]}{14}=\frac{\frac{1}{2}\sdot\left[\left(225+196\right)-169\right]}{14}=\frac{\frac{1}{2}\sdot\left(421-169\right)}{14}\\&\scriptstyle=\frac{\frac{1}{2}\sdot252}{14}=\frac{126}{14}=9\\\end{align}}}](/mediawiki/images/math/3/9/b/39bf1d911e6e3d0c22577ed996e7967e.png)
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle\frac{\frac{1}{2}\sdot\left[\left(AB^2+BG^2\right)-AG^2\right]}{BG}&\scriptstyle=\frac{\frac{1}{2}\sdot\left[\left(13^2+14^2\right)-15^2\right]}{14}=\frac{\frac{1}{2}\sdot\left[\left(169+196\right)-225\right]}{14}=\frac{\frac{1}{2}\sdot\left(365-225\right)}{14}\\&\scriptstyle=\frac{\frac{1}{2}\sdot140}{14}=\frac{70}{14}=5\\\end{align}}}](/mediawiki/images/math/e/b/f/ebffa7c5aa53ccfd67e5875218757ecd.png)

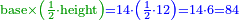


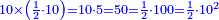
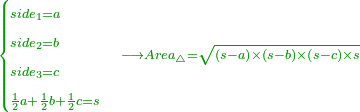

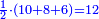
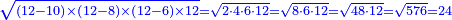
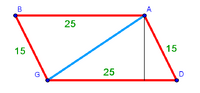
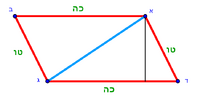

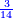 ; the inner circle exceeds its inner square by
; the inner circle exceeds its inner square by 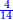 .
.
![\scriptstyle{\color{OliveGreen}{\sqrt{\rm{side}^2-\left[\frac{1}{2}\sdot\left[\left(\rm{bottom\ base}\right)-\left(\rm{upper\ base}\right)\right]\right]^2}}}](/mediawiki/images/math/4/d/d/4dd4d916db1f6b96bf6ff97a571571d7.png)
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle AH&\scriptstyle=\sqrt{AB^2-\left[\frac{1}{2}\sdot\left(BD-AG\right)\right]^2}=\sqrt{13^2-\left[\frac{1}{2}\sdot\left(18-8\right)\right]^2}=\sqrt{169-\left(\frac{1}{2}\sdot10\right)^2}\\&\scriptstyle=\sqrt{169-5^2}=\sqrt{169-25}=\sqrt{144}=12\\\end{align}}}](/mediawiki/images/math/f/2/3/f234251db56850fb612e1a426a4c951a.png)
![\scriptstyle{\color{OliveGreen}{\left[\frac{1}{2}\sdot\left[\left(\rm{top\ base}\right)+\left(\rm{bottom\ base}\right)\right]\right]\sdot\rm{height}}}{\color{blue}{=\left[\frac{1}{2}\sdot\left(8+18\right)\right]\sdot12=\left[\frac{1}{2}\sdot26\right]\sdot12=13\sdot12=156}}](/mediawiki/images/math/f/b/6/fb69e96bf102ceb9d99d5e46825f03a0.png)
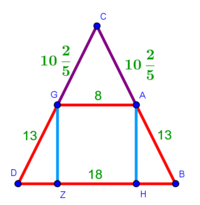

![\scriptstyle{\color{blue}{\begin{align}\scriptstyle AC:13=AC:BC=GC:13&\scriptstyle=GC:DC=AG:\left(BD-AG\right)\\&\scriptstyle={\color{OliveGreen}{\left(\rm{top\ base}\right):\left[\left(\rm{bottom\ base}\right)-\left(\rm{top\ base}\right)\right]}}\\&\scriptstyle=8:\left(18-8\right)=8:10=\frac{4}{5}=1-\frac{1}{5}\\\end{align}}}](/mediawiki/images/math/0/c/4/0c4d197b6c60509ca901c349a7530857.png)
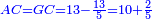
![\scriptstyle{\color{OliveGreen}{\rm{height}\sdot\left[\frac{1}{2}\sdot\left[\left(\rm{top\ base}\right)+\left(\rm{bottom\ base}\right)\right]\right]}}](/mediawiki/images/math/5/b/5/5b521955d63ba10eb7311e9a47d6fe46.png)
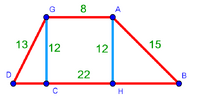
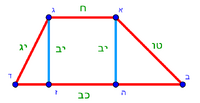
![\scriptstyle{\color{blue}{12\sdot\left[\frac{1}{2}\sdot\left(8+22\right)\right]=12\sdot\left(\frac{1}{2}\sdot30\right)=12\sdot15=180}}](/mediawiki/images/math/d/6/d/d6d1aad37c46030ca055b78ae6edd836.png)
![\scriptstyle{\color{OliveGreen}{height\sdot\left[\frac{1}{2}\sdot\left[\left(top\ base\right)+\left(bottom\ base\right)\right]\right]}}](/mediawiki/images/math/b/1/d/b1df4ea4bb7e3bb8fba4815bd773d7dc.png)
![\scriptstyle{\color{blue}{9\sdot\left[\frac{1}{2}\sdot\left(8+20\right)\right]=9\sdot\left(\frac{1}{2}\sdot28\right)=9\sdot14=126}}](/mediawiki/images/math/6/8/b/68b1007c14e93b43f21b6fecffbb9f64.png)
![\scriptstyle{\color{blue}{12\sdot\left[\frac{1}{2}\sdot\left(14+21\right)\right]=12\sdot\left(17+\frac{1}{2}\right)=210}}](/mediawiki/images/math/5/0/f/50f1b9c85f19e17260ef8843108adc68.png)

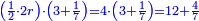
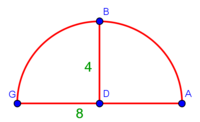
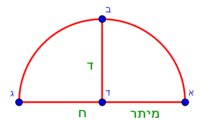
![\scriptstyle{\color{blue}{4\sdot\left[\frac{1}{2}\sdot\left(12+\frac{4}{7}\right)\right]=4\sdot\left(6+\frac{2}{7}\right)=25+\frac{1}{7}}}](/mediawiki/images/math/7/6/4/764622b2cd768983aec6d8c70c988879.png)
![\scriptstyle{\color{OliveGreen}{\frac{1}{2}\sdot\left[\left(2r\right)^2-\left[\left[\frac{1}{7}+\left(\frac{1}{2}\sdot\frac{1}{7}\right)\right]\sdot\left(2r\right)^2\right]\right]}}](/mediawiki/images/math/6/b/6/6b6e41a83d19b7fa663f7310300898cb.png)
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle\frac{1}{2}\sdot\left[8^2-\left[\left[\frac{1}{7}+\left(\frac{1}{2}\sdot\frac{1}{7}\right)\right]\sdot8^2\right]\right]&\scriptstyle=\frac{1}{2}\sdot\left[64-\left[\left[\frac{1}{7}+\left(\frac{1}{2}\sdot\frac{1}{7}\right)\right]\sdot64\right]\right]=\frac{1}{2}\sdot\left[64-\left(13+\frac{5}{7}\right)\right]\\&\scriptstyle=\frac{1}{2}\sdot\left(50+\frac{2}{7}\right)=25+\frac{1}{7}\\\end{align}}}](/mediawiki/images/math/b/9/a/b9a4238e71f7081b6fab96916d3c3a15.png)
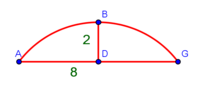
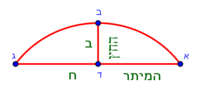
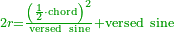


![\scriptstyle{\color{blue}{ABGD=AG_{sector}-ACG_{\triangle}=AH\sdot\left(\frac{1}{2}\sdot AG_{arc}\right)-ACG_{\triangle}=\left[r\sdot\left(\frac{1}{2}\sdot AG_{arc}\right)\right]-ACG_{\triangle}}}](/mediawiki/images/math/c/3/3/c331f26055d92bc081ccfe8c2793bc17.png)
![\scriptstyle{\color{OliveGreen}{\left[\left[\frac{1}{2}\sdot\left(2r\right)\right]\sdot\left(\frac{1}{2}\sdot\rm{arc}\right)\right]-\left[\left[\left[\frac{1}{2}\sdot\left(2r\right)\right]-\rm{versed\ sine}\right]\sdot\left(\frac{1}{2}\sdot\rm{chord}\right)\right]}}](/mediawiki/images/math/d/6/0/d605d4f78e9bef02470d63d9544f369b.png)


![\scriptstyle{\color{OliveGreen}{\left[\left[\frac{1}{2}\sdot\left(2r\right)\right]\sdot\left(\frac{1}{2}\sdot\rm{arc}\right)\right]+\rm{Area}_{\triangle}}}](/mediawiki/images/math/d/1/6/d16d06efbeaef35e417b2c19bff017b0.png)
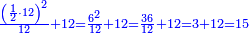
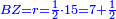

![\scriptstyle{\color{blue}{ABGDA=ABGZA+AZG=ABGZA+\left[DZ\sdot\left(\frac{1}{2}\sdot AG\right)\right]}}](/mediawiki/images/math/d/7/a/d7a5b97626d14c9dc6d3d9b48ea613a6.png)
![\scriptstyle{\color{OliveGreen}{\left[\left[\frac{1}{2}\sdot\left(2r\right)\right]\sdot\left(\frac{1}{2}\sdot\rm{arc}\right)\right]+\left[\left[\rm{versed\ sine}-\left[\frac{1}{2}\sdot\left(2r\right)\right]\right]\sdot\left(\frac{1}{2}\sdot\rm{chord}\right)\right]}}](/mediawiki/images/math/6/d/4/6d432194c21ae92fef81e69b723c15a3.png)

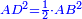
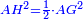
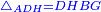
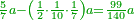
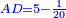


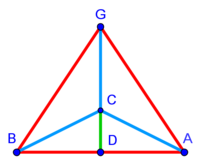
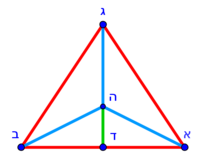
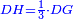
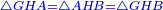





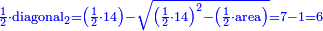
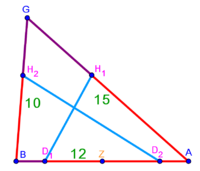
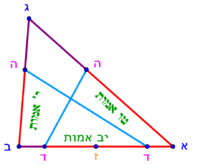
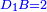
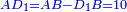
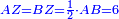
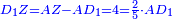

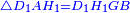

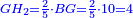
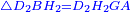




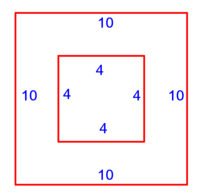

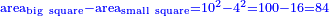


![\scriptstyle{\color{OliveGreen}{\left[\frac{1}{2}\sdot\left(\rm{base_{bottom}-base_{top}}\right)\right]+\frac{\frac{1}{2}\sdot\left(\rm{side_1^2-side_2^2}\right)}{\rm{base_{bottom}-base_{top}}}}}](/mediawiki/images/math/8/c/9/8c9b6d1d38374842b7d95e80879134c9.png)
![\scriptstyle{\color{OliveGreen}{\left[\frac{1}{2}\sdot\left(\rm{base_{bottom}-base_{top}}\right)\right]-\frac{\frac{1}{2}\sdot\left(\rm{side_1^2-side_2^2}\right)}{\rm{base_{bottom}-base_{top}}}}}](/mediawiki/images/math/a/b/0/ab088396870fa8692f26053d4809f487.png)
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle BH &\scriptstyle=\left[\frac{1}{2}\sdot\left(BD-AG\right)\right]+\frac{\frac{1}{2}\sdot\left(AB^2-GD^2\right)}{BD-AG}=\left[\frac{1}{2}\sdot\left(22-8\right)\right]+\frac{\frac{1}{2}\sdot\left(15^2-13^2\right)}{22-8}\\&\scriptstyle=\left(\frac{1}{2}\sdot14\right)+\frac{\frac{1}{2}\sdot\left(15^2-169\right)}{14}=7+\frac{\frac{1}{2}\sdot56}{14}=7+\frac{28}{14}=7+2=9\\\end{align}}}](/mediawiki/images/math/a/7/8/a7855f2dfb8394a9f6ed7785513f77d2.png)
![\scriptstyle{\color{blue}{CD=\left[\frac{1}{2}\sdot\left(BD-AG\right)\right]-\frac{\frac{1}{2}\sdot\left(AB^2-GD^2\right)}{BD-AG}=7-2}}](/mediawiki/images/math/1/0/2/1027a9c6e067c0548a875e01e5376d46.png)
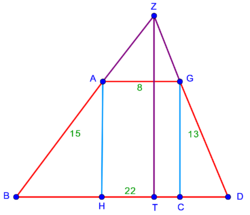

![\scriptstyle{\color{blue}{AZ=\frac{HT\sdot AB}{HB}=\frac{\left(BT-HB\right)\sdot AB}{HB}=\frac{\left[\left(14+\frac{1}{7}\right)-9\right]\sdot 15}{9}=\frac{\left(5+\frac{1}{7}\right)\sdot15}{9}=\frac{77+\frac{1}{7}}{9}=8+\frac{4}{7}}}](/mediawiki/images/math/5/a/8/5a8bdc31cf4a115ef3b3e6869789c4e0.png)
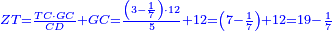
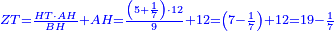

![\scriptstyle{\color{OliveGreen}{\left[\frac{1}{2}\sdot\left(\rm{base_{top}+base_{bottom}}\right)\right]\sdot\rm{height}}}](/mediawiki/images/math/0/a/4/0a462b7f7ae6f829c66786fc98113e09.png)
![\scriptstyle{\color{blue}{\left[\frac{1}{2}\sdot\left(8+20\right)\right]\sdot9=\left(\frac{1}{2}\sdot28\right)\sdot9=14\sdot9=126}}](/mediawiki/images/math/8/a/2/8a2be587e347ec5a64296a52ea860b9f.png)



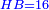
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle CD &\scriptstyle=\frac{\frac{1}{2}\sdot\left[AB^2-\left[\left(BD-AG\right)^2+GD^2\right]\right]}{BD-AG}=\frac{\frac{1}{2}\sdot\left[20^2-\left[\left(21-14\right)^2+15^2\right]\right]}{21-14}=\frac{\frac{1}{2}\sdot\left[20^2-\left(7^2+15^2\right)\right]}{7}\\&\scriptstyle=\frac{\frac{1}{2}\sdot\left(400-274\right)}{7}=\frac{\frac{1}{2}\sdot126}{7}=\frac{63}{7}=9\\\end{align}}}](/mediawiki/images/math/b/2/8/b28bbc6bd98c9e3890aa1105e145e1d1.png)
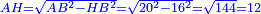

![\scriptstyle{\color{blue}{\left[\frac{1}{2}\sdot\left(14+21\right)\right]\sdot12=210}}](/mediawiki/images/math/8/c/b/8cb8b983e66e5f5777b2663053b8e239.png)

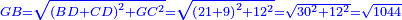


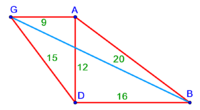
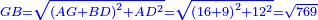
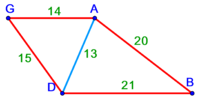
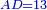
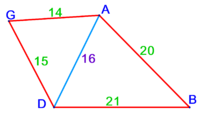
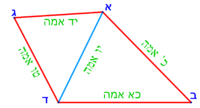
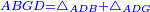
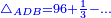
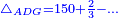
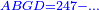
![\scriptstyle{\color{OliveGreen}{\left[\frac{1}{2}\sdot\left(\rm{height_1+height_2}\right)\right]\sdot\rm{diagonal}}}](/mediawiki/images/math/3/1/1/311d7ab92aed0202cb3874127b6a5a58.png)

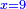

![\scriptstyle{\color{blue}{x=\frac{1}{2}\sdot\left(10+\frac{5^2}{10}\right)=\frac{1}{2}\sdot\left(10+\frac{25}{10}\right)=\frac{1}{2}\sdot\left[10+\left(2+\frac{1}{2}\right)\right]=6+\frac{1}{4}}}](/mediawiki/images/math/2/1/f/21f4dd1709c59b361a8076494299eed2.png)
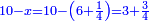
![\scriptstyle1+\left[\frac{1}{10}\sdot\left(X-1\right)\right]=2+\frac{1}{10}\sdot\left[X-\left[\left[1+\left[\frac{1}{10}\sdot\left(X-1\right)\right]\right]+2\right]\right]](/mediawiki/images/math/0/4/e/04ec9fa2f7109a05d7c4e8fa7ce5d879.png)