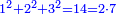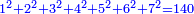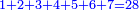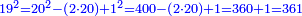Difference between revisions of "ספר האחד"
From mispar
(→One) |
(→Appendix: Bibliography) |
||
| (49 intermediate revisions by 2 users not shown) | |||
| Line 1: | Line 1: | ||
| − | {{#annotpage: author="Abraham ben Meir Ibn ‛Ezra", city="Béziers", | + | {{#annotpage: author="Abraham ben Meir Ibn ‛Ezra", city="Béziers", time="1148"}} |
{| | {| | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|‫<ref>37</ref>בשם האחד לבדו יחל אברהם עבדו | ||
|- | |- | ||
| | | | ||
| Line 8: | Line 11: | ||
| | | | ||
*The one counts itself and no other counts it, rather it counts every number. | *The one counts itself and no other counts it, rather it counts every number. | ||
| − | |style="text-align:right;"|האחד סופר עצמו ואין אחר סופרו<ref>[[ | + | |style="width: 50%; text-align:right;"|האחד סופר את עצמו ואין אחר סופרו‫<ref>[[פירוש_ספר_האחד_/_מרדכי_כומטינו#האחד_סופר_את_עצמו_ואין_אחר_סופרו|האחד סופר את עצמו ואין אחר סופרו]]</ref> והוא יספור כל מספר‫<ref>[[פירוש_ספר_האחד_/_מרדכי_כומטינו#והוא_כל_מספר|והוא סופר כל מספר]]</ref> |
|- | |- | ||
| | | | ||
*It is a square root, a cube root, a square and a cube. | *It is a square root, a cube root, a square and a cube. | ||
| − | |style="text-align:right;"|והוא שרש ויסוד ומרבע ומעקב | + | |style="text-align:right;"|והוא שורש ויסוד ומרובע ומעוקב‫<ref>[[פירוש_ספר_האחד_/_מרדכי_כומטינו#והוא_שרש_ויסוד_ומרבע_ומעקב|והוא שרש ויסוד ומרבע ומעקב]]</ref> |
|- | |- | ||
| | | | ||
*It resembles the substance of the thing that bears the accidents. | *It resembles the substance of the thing that bears the accidents. | ||
| − | |style="text-align:right;"|והוא דומה לעצם הדבר הנושא כל המקרים | + | |style="text-align:right;"|והוא דומה לעצם הדבר הנושא כל המקרים‫<ref>[[פירוש_ספר_האחד_/_מרדכי_כומטינו#והוא_דומה_לעצם_הדבר_הנושא_כל_המקרים|והוא דומה לעצם הדבר הנושא כל המקרים]]</ref> |
|- | |- | ||
| | | | ||
*Every number is in its potentiality and it is in every number in practice. | *Every number is in its potentiality and it is in every number in practice. | ||
| − | |style="text-align:right;"|וכל מספר בכחו והוא בכל מספר במעשה | + | |style="text-align:right;"|וכל מספר בכחו<ref>marg. וכל מספר הוא בו בכח</ref> והוא בכל מספר במעשה‫<ref>[[פירוש_ספר_האחד_/_מרדכי_כומטינו#וכל_מספר_בכחו_והוא_בכל_מספר_במעשה|וכל מספר בכחו והוא בכל מספר במעשה]]</ref> |
|- | |- | ||
| | | | ||
*It is the existence and every number exists for it. | *It is the existence and every number exists for it. | ||
| − | |style="text-align:right;"|והוא ההוה וכל מספר הוה בעבורו | + | |style="text-align:right;"|והוא ההוה וכל מספר הוה בעבורו‫<ref>[[פירוש_ספר_האחד_/_מרדכי_כומטינו#והוא_ההוה_וכל_מספר_הוה_בעבורו|והוא ההוה וכל מספר הוה בעבורו]]</ref> |
|- | |- | ||
| | | | ||
*It is eternal and every number is created. | *It is eternal and every number is created. | ||
| − | |style="text-align:right;"|והוא קדמון וכל מספר מתחדש | + | |style="text-align:right;"|והוא קדמון לכל וכל מספר מתחדש‫<ref>[[פירוש_ספר_האחד_/_מרדכי_כומטינו#והוא קדמון_וכל_מספר_מתחדש|והוא קדמון וכל מספר מתחדש]]</ref> |
|- | |- | ||
| | | | ||
| Line 36: | Line 39: | ||
| | | | ||
*It is not a number. | *It is not a number. | ||
| − | |style="text-align:right;"|והוא אינו מספר | + | |style="text-align:right;"|והוא אינו מספר‫<ref>[[פירוש_ספר_האחד_/_מרדכי_כומטינו#והוא_סבת_כל_מספר_זוג_ושאינו_זוג_והוא_אינו_מספר|והוא סבת כל מספר זוג ושאינו זוג והוא אינו מספר]]</ref> |
|- | |- | ||
| | | | ||
*It is not multiplied or divided, but is the cause of all multiplication and division. | *It is not multiplied or divided, but is the cause of all multiplication and division. | ||
| − | |style="text-align:right;"|ולא ירבה ולא יתחלק והוא סבת כל רבוי וחלוק | + | |style="text-align:right;"|ולא ירבה ולא יתחלק והוא סבת כל רבוי וחילוק‫<ref>[[פירוש_ספר_האחד_/_מרדכי_כומטינו#ולא_ירבה_ולא_יחלק_והוא_סבת_כל_רבוי_וחלוק|ולא ירבה ולא יחלק והוא סבת כל רבוי וחלוק]]</ref> |
|- | |- | ||
| | | | ||
*It has no similarity, no opposite and no alteration. | *It has no similarity, no opposite and no alteration. | ||
| − | |style="text-align:right;"|ואין לו דמיון גם אין לו הפך ולא שנוי | + | |style="text-align:right;"|ואין לו דמיון גם אין לו הפך ולא שינוי‫<ref>[[פירוש_ספר_האחד_/_מרדכי_כומטינו#ואין_לו_שנוי|ואין לו שנוי]]</ref> |
|- | |- | ||
| | | | ||
*It does with one side what every number does with two sides. | *It does with one side what every number does with two sides. | ||
| − | |style="text-align:right;"|והוא יעשה בפאה אחת מה שיעשה כל חשבון בשתי פאות | + | |style="text-align:right;"|והוא יעשה בפאה אחת מה שיעשה כל חשבון בשתי פאות‫<ref>[[פירוש_ספר_האחד_/_מרדכי_כומטינו#והוא_יעשה_בפאה_אחת_מה_שעושה_כל_חשבון_בשתי_פאות|והוא יעשה בפאה אחת מה שעושה כל חשבון בשתי פאות]]</ref> |
|- | |- | ||
| | | | ||
*It encompasses every whole and every part, because it is the first and the last; it has no fraction, only in the thought of dividing the whole so that it will become one. | *It encompasses every whole and every part, because it is the first and the last; it has no fraction, only in the thought of dividing the whole so that it will become one. | ||
| − | |style="text-align:right;"|והוא כולל כל כלל וכל חלק כי הוא ראשון ואחרון ואין לו שבר כי אם במחשבת | + | |style="text-align:right;"|והוא כולל כל כלל וכל חלק כי הוא ראשון‫<ref>[[פירוש_ספר_האחד_/_מרדכי_כומטינו#והוא_כולל_כל_כלל_וכל_חלק_כי_הוא_ראשון|והוא כולל כל כלל וכל חלק כי הוא ראשון]]</ref> ואחרון ואין לו שבר‫<ref>[[פירוש_ספר_האחד_/_מרדכי_כומטינו#אין_לו_שבר|אין לו שבר]]</ref> כי אם במחשבת חלק הכלל שיהיה אחד |
|- | |- | ||
| | | | ||
*It is the beginning of all the odd numbers that generate the square numbers when summed successively. | *It is the beginning of all the odd numbers that generate the square numbers when summed successively. | ||
| − | |style="text-align:right;"|והוא תחלת כל מספר שאינו זוג | + | |style="text-align:right;"|והוא תחלת כל מספר שאינו זוג המחוברים‫<ref>המחוברים marg. נ' למרובעים</ref> על סדר המולידים המרובעים‫<ref>[[פירוש_ספר_האחד_/_מרדכי_כומטינו#והוא_תחלת_כל_מספר_שאינו_זוג_המחברי'_על_סדר_המולידי'_המרבעי'|והוא תחלת כל מספר שאינו זוג המחברי' על סדר המולידי' המרבעי']]</ref> |
|- | |- | ||
| | | | ||
*It is also the beginning of all the even numbers, where the products of the extremes are equal to one another | *It is also the beginning of all the even numbers, where the products of the extremes are equal to one another | ||
| − | |style="text-align:right;"|גם הוא תחלת כל זוג שתהיינה הקצוות כפל זה על זה שוות | + | |style="text-align:right;"|גם הוא תחלת כל זוג שתהיינה הקצוות כפל זה על זה שוות‫<ref>[[פירוש_ספר_האחד_/_מרדכי_כומטינו#גם_הוא_תחלת_כל_זוג_שתהיינה_הקצוו'_כפל_זה_על_זה_שוות|גם הוא תחלת כל זוג שתהיינה הקצוו' כפל זה על זה]]</ref> |
|- | |- | ||
| | | | ||
:and if the number is odd, the product is as the square of the mean. | :and if the number is odd, the product is as the square of the mean. | ||
| − | |style="text-align:right;"|ואם המספר איננו זוג יהיה הכפל | + | |style="text-align:right;"|ואם המספר איננו זוג יהיה הכפל ומרובע האמצעי‫<ref>[[פירוש_ספר_האחד_/_מרדכי_כומטינו#ואם_המספר_איננו_זוג_יהיה_הכפל_כמרבעי_האמצעי|ואם המספר איננו זה יהיה הכפל כמרבעי האמצעי]]</ref> |
|- | |- | ||
| | | | ||
:and the difference between each square and square is as one part and half of the part. | :and the difference between each square and square is as one part and half of the part. | ||
| − | |style="text-align:right;"|ובין מרבע למרבע דמות חלק וחצי | + | |style="text-align:right;"|ובין מרובע למרובע דמות חלק וחצי חלק‫<ref>[[פירוש_ספר_האחד_/_מרדכי_כומטינו#ובין_מרבע_למרבע_דמות_חלק_וחצי_החלק|ובין מרבע למרבע דמות חלק וחצי החלק]]</ref> |
|- | |- | ||
| | | | ||
*Since the one is not a fraction, the two are parts in the right sense, and any [kind of] parts is like the two. | *Since the one is not a fraction, the two are parts in the right sense, and any [kind of] parts is like the two. | ||
| − | |style="text-align:right;"|ובעבור | + | |style="text-align:right;"|ובעבור שאחד אינו שבור היו השנים חלקים נכונים‫<ref>[[פירוש_ספר_האחד_/_מרדכי_כומטינו#ובעבור_שהאחד_איננו_שבור_היו_השני'_חלקי_נכוני'|ובעבור שהאחד איננו שבור היו השני' חלקי נכוני']]</ref> וכן כל חלק וחלק כשנים‫<ref>[[פירוש_ספר_האחד_/_מרדכי_כומטינו#וכן_כל_חלק_וחלק_בשנים|וכן כל חלק וחלק בשנים]]</ref> |
|- | |- | ||
| | | | ||
*For every triangular shape that is the beginning of the shapes and every shape is divided into it: | *For every triangular shape that is the beginning of the shapes and every shape is divided into it: | ||
| − | |style="text-align:right;"|וכל צורה | + | |style="text-align:right;"|וכל צורה משולשת שהיא תחלת הצורות ועליה תתחלק כל הצורה‫<ref>[[פירוש_ספר_האחד_/_מרדכי_כומטינו#וכל_צורה_משלשת|וכל צורה משלשת]]</ref> |
|- | |- | ||
| | | | ||
:If one side [of the shape] is one, the triangle can only be equilateral or isosceles acute triangle, because then the base have to be one. | :If one side [of the shape] is one, the triangle can only be equilateral or isosceles acute triangle, because then the base have to be one. | ||
| − | |style="text-align:right;"|ואם | + | |style="text-align:right;"|ואם היה הצלע האחר ג' אחד לא יתכן להיות המשולש כי ‫<ref>38r</ref>אם שוה כולו או שוה השוקים רק חדי המקצוע כי המכונה תהיה אחת |
|- | |- | ||
| | | | ||
*And the number ten is analogous to one, as are the hundred and thousand successively. | *And the number ten is analogous to one, as are the hundred and thousand successively. | ||
| − | |style="text-align:right;"|וחשבון עשרה דומה לאחד וככה מאה ואלף על הסדר | + | |style="text-align:right;"|וחשבון עשרה דומה לאחת וככה מאה ואלף על הסדר‫<ref>[[פירוש_ספר_האחד_/_מרדכי_כומטינו#וחשבון_עשרה_דומה_לאחד_וככה_מאה_ואלף_על_הסדר|וחשבון עשרה דומה לאחד וככה מאה ואלף על הסדר]]</ref> |
|- | |- | ||
| | | | ||
*And the arithmeticians, when they want to multiply a number by a number in one or two ranks, they subtract one for the foundation; and they gave many reasons for this. | *And the arithmeticians, when they want to multiply a number by a number in one or two ranks, they subtract one for the foundation; and they gave many reasons for this. | ||
| − | |style="text-align:right;"|וחכמי המספר ברצותם | + | |style="text-align:right;"|וחכמי המספר ברצותם לכפול חשבון על חשבון במערכה אחת או בשתים יחסרו אחד למוסד והאריכו לתת טעם |
|- | |- | ||
| | | | ||
:But, the principle is that the analogy begins in the second rank. | :But, the principle is that the analogy begins in the second rank. | ||
| − | |style="text-align:right;"|והעקר כי | + | |style="text-align:right;"|והעקר כי המערכת השנית שם יחל הדמיון |
|- | |- | ||
| | | | ||
:And to make it easier for the students, they calculated in the first rank, which are the units and added for the two numbers. Therefore, they had to subtract one. | :And to make it easier for the students, they calculated in the first rank, which are the units and added for the two numbers. Therefore, they had to subtract one. | ||
| − | |style="text-align:right;"|ובעבור | + | |style="text-align:right;"|ובעבור הקל על התלמידים חשבו המערכת הראשונה שהם האחדים והוסיפו השנים על השנים כמספרם על כן הוצרכו לחסר אחת |
|- | |- | ||
| | | | ||
:Because the rank of the tens is really one, the hundreds two, the thousands three, so the reason is the same. | :Because the rank of the tens is really one, the hundreds two, the thousands three, so the reason is the same. | ||
| − | |style="text-align:right;"|כי מערכת העשרות באמת | + | |style="text-align:right;"|כי מערכת העשרות באמת אחת והמאות שנים והאלפים שלשה והדבר שוה |
|- | |- | ||
| | | | ||
| Line 113: | Line 116: | ||
| | | | ||
*It is called so, because it receives a difference, as the sentence: ''And their laws differ from [those of] every people'' [Esther 3, 8]. | *It is called so, because it receives a difference, as the sentence: ''And their laws differ from [those of] every people'' [Esther 3, 8]. | ||
| − | |style="text-align:right;"|ונקרא כן בעבור | + | |style="text-align:right;"|ונקרא כן בעבור שהוא מקבל שינוי מגזרת ''ודתיהם שונות''‫<ref>אסתר, ג, ח</ref> |
|- | |- | ||
| | | | ||
| Line 121: | Line 124: | ||
| | | | ||
*Every being is substance and form, but the One is not so. | *Every being is substance and form, but the One is not so. | ||
| − | |style="text-align:right;"|וכל יש עצם וצורה ולא כן האחד | + | |style="text-align:right;"|וכל [יש]<ref>om.</ref> עצם וצורה ולא כן האחד |
|- | |- | ||
| | | | ||
*Every triangle, whose one side is two, can only be either equilateral or isosceles or obtuse. | *Every triangle, whose one side is two, can only be either equilateral or isosceles or obtuse. | ||
| − | |style="text-align:right;"|וכל | + | |style="text-align:right;"|וכל משולש שתהא הצלע האחת שתים ולא יתכן להיותו כי אם שוה כולו או שוקיו שוות או המקצוע רחב |
|- | |- | ||
| | | | ||
*Know that all triangles are divided into two different categories: | *Know that all triangles are divided into two different categories: | ||
| − | |style="text-align:right;"|ודע כי כל | + | |style="text-align:right;"|ודע כי כל משולש מתחלק לשנים חלקים שו<sup>נ</sup>ים |
|- | |- | ||
| | | | ||
:*The one is uniform and it is in two ways: equilateral or isosceles, all angles of which are always acute. | :*The one is uniform and it is in two ways: equilateral or isosceles, all angles of which are always acute. | ||
| − | |style="text-align:right;"|האחד שוה והוא על שנים דרכים שוה | + | |style="text-align:right;"|האחד שוה והוא על שנים דרכים שוה כולו או שתי שוקיו ולעולם יהיו כל המקצועים חדים |
|- | |- | ||
| | | | ||
:*The second category: whose sides are unequal and it is in three ways: | :*The second category: whose sides are unequal and it is in three ways: | ||
| − | |style="text-align:right;"|והחלק השני | + | |style="text-align:right;"|והחלק השני תהיינה הצלעות משתנות והוא על שלשה דרכים |
|- | |- | ||
| | | | ||
::*The one whose one angle is obtuse, because two angles are always acute. | ::*The one whose one angle is obtuse, because two angles are always acute. | ||
| − | |style="text-align:right;"|האחד שיהיה המקצוע רחב כי | + | |style="text-align:right;"|האחד שיהיה המקצוע רחב כי שתי המקצועות לעולם חדים |
|- | |- | ||
| | | | ||
:::The square of the largest side exceeds the sum of the squares of the two smaller sides by double the product of one [of the smaller] sides multiplied by its own extension up to its height that falls on the outside. | :::The square of the largest side exceeds the sum of the squares of the two smaller sides by double the product of one [of the smaller] sides multiplied by its own extension up to its height that falls on the outside. | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ומרובע הצלע הגדול הוא מוסיף על שני מרובעי הצלעות [הקטנות]‫<ref>om.</ref> ככפל אחד חלקי קו הצלע על החלק השני עם התוספת על הצלע עד היותה לנוכח העמוד שהוא מבחוץ |
|- | |- | ||
| | | | ||
:::The beginning of the obtuse-angled triangles is by the succession: 2, 3, 4. | :::The beginning of the obtuse-angled triangles is by the succession: 2, 3, 4. | ||
| − | |style="text-align:right;"|ותחלת כל | + | |style="text-align:right;"|ותחלת כל משולש רחב המקצוע על הסדר בג"ד |
|- | |- | ||
| | | | ||
::*The second type is where one angle is a right angle and the [other] two are also acute. | ::*The second type is where one angle is a right angle and the [other] two are also acute. | ||
| − | |style="text-align:right;"|והדרך השני להיות המקצוע האחד ישר גם השנים | + | |style="text-align:right;"|והדרך השני להיות המקצוע האחד ישר גם השנים מקצועות יהיו חדים |
|- | |- | ||
| | | | ||
:::The [sum of the] squares of the two smaller [sides] is as the square of the hypotenuse, which is the largest side. | :::The [sum of the] squares of the two smaller [sides] is as the square of the hypotenuse, which is the largest side. | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ומרובעי השנים הקטנות כמרובע היתר שהוא הצלע הגדול |
|- | |- | ||
| | | | ||
::*The third type is called acute-angled, because all of its angles are acute. | ::*The third type is called acute-angled, because all of its angles are acute. | ||
| − | |style="text-align:right;"|והדרך השלישי יקרא חד המקצוע כי כל מקצועותיו חדים | + | |style="text-align:right;"|והדרך השלישי יקרא חד‫<ref>L: אחד</ref> המקצוע כי כל מקצועותיו חדים |
|- | |- | ||
| | | | ||
| Line 170: | Line 173: | ||
| | | | ||
*The three is opposite to the one, because the sum of one with one is greater than its product by itself, the calculation of two is equal, and the sum of three with three is smaller than its product by itself. The way of three applies to every [other] number. | *The three is opposite to the one, because the sum of one with one is greater than its product by itself, the calculation of two is equal, and the sum of three with three is smaller than its product by itself. The way of three applies to every [other] number. | ||
| − | |style="text-align:right;"|השלשה הפך האחד כי מחברת אחד אל אחד גדול מכפלו בעצמו וחשבון השנים שוה ומחברת | + | |style="text-align:right;"|השלשה הפך האחד כי מחברת אחד אל אחד גדול מכפלו בעצמו וחשבון השנים שוה ומחברת ג' אל ג' קטן מכפלו בעצמו ועל דרך שלשה הוא בכל חשבון |
|- | |- | ||
| | | | ||
*It is the beginning of the triangular numbers. | *It is the beginning of the triangular numbers. | ||
| − | |style="text-align:right;"|והוא תחלת מספר | + | |style="text-align:right;"|והוא תחלת מספר משולש שוה |
|- | |- | ||
| | | | ||
*Its square is the number that is the beginning of the [scalene] triangles. | *Its square is the number that is the beginning of the [scalene] triangles. | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ומרובעו כמספר תחלת משולש [בלתי שוה]‫<ref>L: שהיה</ref> |
|- | |- | ||
| | | | ||
*Its cube is the number of sides of the equilateral triangles that are in the first rank. | *Its cube is the number of sides of the equilateral triangles that are in the first rank. | ||
| − | |style="text-align:right;"|ומעקבו מספר צלעות השוה שהם במערכת הראשונה | + | |style="text-align:right;"|ומעקבו‫<ref>L: וכמו מעוקבו</ref> מספר צלעות השוה שהם במערכת הראשונה |
|- | |- | ||
| | | | ||
*The number three is the beginning of every number that is not even [= odd numbers], whose product by itself is greater than itself. | *The number three is the beginning of every number that is not even [= odd numbers], whose product by itself is greater than itself. | ||
| − | |style="text-align:right;"|ומספר | + | |style="text-align:right;"|ומספר שלשה תחלת כל מספר שאיננו זוג וכפלו על עצמו גדול ממנו |
|- | |- | ||
| | | | ||
*There is no number other than it, such that the sum of the preceding numbers is the same as itself. | *There is no number other than it, such that the sum of the preceding numbers is the same as itself. | ||
| − | |style="text-align:right;"|ואין מספר | + | |style="text-align:right;"|ואין מספר שיהיה המספרים לפניו כמותו רק הוא לבדו |
|- | |- | ||
| | | | ||
:Therefore, the number six that consists of it is equal [to the sum of] its parts. | :Therefore, the number six that consists of it is equal [to the sum of] its parts. | ||
| − | |style="text-align:right;"|על כן היה חשבון ששה שהוא | + | |style="text-align:right;"|על כן היה חשבון ששה שהוא מורכב ממנו ישר עם חלקיו |
|- | |- | ||
| | | | ||
| − | :The number that consists of the | + | :The number that consists of the three is the beginning of the numbers whose parts exceed over them. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והמורכב מהשלשה תחלת <sup>ה</sup>חשבון שחלקיו רבים ממנו |
|- | |- | ||
| | | | ||
*If you sum it with its parts, the sum is a perfect number. | *If you sum it with its parts, the sum is a perfect number. | ||
| − | |style="text-align:right;"|ואם תחברנו עם חלקיו יהיה | + | |style="text-align:right;"|ואם תחברנו עם חלקיו יהיה מספר המחובר חשבון ישר |
|- | |- | ||
| | | | ||
*Know that the one is as the length, which is the line. | *Know that the one is as the length, which is the line. | ||
| − | |style="text-align:right;"|ודע כי האחד כמו | + | |style="text-align:right;"|ודע כי האחד כמו אורך שהוא קו |
|- | |- | ||
| | | | ||
:Two is as the surface | :Two is as the surface | ||
| − | |style="text-align:right;"|והשנים שטח | + | |style="text-align:right;"|‫[והשנים שטח]‫<ref>L om.</ref> |
|- | |- | ||
| | | | ||
:And the three is as the body, for it has length, breadth and depth. | :And the three is as the body, for it has length, breadth and depth. | ||
| − | |style="text-align:right;"|והשלשה גוף כי יש לו אֹרך ורֹחב | + | |style="text-align:right;"|‫[והשלשה]‫<ref>L: והשלשה והשנים</ref> גוף כי יש לו אֹרך ורֹחב ועומק |
|- | |- | ||
| | | | ||
*The theologians say that the time is divided into three. | *The theologians say that the time is divided into three. | ||
| − | |style="text-align:right;"|ואמרו חכמי | + | |style="text-align:right;"|ואמרו חכמי התושייה כי הזמן מתחלק על שלשה |
|- | |- | ||
| | | | ||
*The vegetative soul, the vital soul and the rational soul are three. | *The vegetative soul, the vital soul and the rational soul are three. | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|הנפש הצומחת והחיה והחכמה שלש |
|- | |- | ||
| | | | ||
| Line 235: | Line 238: | ||
| | | | ||
*The four is the first visible square. | *The four is the first visible square. | ||
| − | |style="text-align:right;"|הארבעה | + | |style="text-align:right;"|הארבעה מרובע ראשון נראה |
|- | |- | ||
| | | | ||
*It is the beginning of the even-times-even numbers. | *It is the beginning of the even-times-even numbers. | ||
| − | |style="text-align:right;"|והוא תחלת | + | |style="text-align:right;"|והוא תחלת החשבון זוג הזוג |
|- | |- | ||
| | | | ||
*It is the beginning of the composite numbers. | *It is the beginning of the composite numbers. | ||
| − | |style="text-align:right;"|והוא תחלת | + | |style="text-align:right;"|והוא תחלת החשבון מורכב |
|- | |- | ||
| | | | ||
:For the first rank are 9 [numbers], of which the one is the foundation of all numbers, the four prime numbers remain: 2, 3, 5, 7; and the other four are composed. | :For the first rank are 9 [numbers], of which the one is the foundation of all numbers, the four prime numbers remain: 2, 3, 5, 7; and the other four are composed. | ||
| − | |style="text-align:right;"|כי מערכת | + | |style="text-align:right;"|כי מערכת הראשונה הם ט' והנה האחד יסוד החשבון ונשארו ארבעה ראשונים והם בגה"ז והארבעה האחרים הם מורכבים |
|- | |- | ||
| | | | ||
*With the sum of the preceding numbers they are ten, which is the beginning of the analogue decade, and four is the principle. | *With the sum of the preceding numbers they are ten, which is the beginning of the analogue decade, and four is the principle. | ||
| − | |style="text-align:right;"|ובחשבון המספרים לפניו הם עשרה שהוא תחלת הכלל הדומה | + | |style="text-align:right;"|ובחשבון המספרים לפניו הם עשרה שהוא תחלת הכלל הדומה והשורש ד‫' |
|- | |- | ||
| | | | ||
| Line 259: | Line 262: | ||
| | | | ||
:Likewise 2 with 5, 3 with 6, 4 with 7 and so on endlessly. | :Likewise 2 with 5, 3 with 6, 4 with 7 and so on endlessly. | ||
| − | |style="text-align:right;"|וככה ב' עם ה' וג' עם ו' וד' עם ז' וככה עד אין קץ | + | |style="text-align:right;"|וככה ב' עם ה' וג' עם ו' וד' עם ז' וככה ‫<ref>38v</ref>עד אין קץ |
|- | |- | ||
| | | | ||
| Line 295: | Line 298: | ||
| | | | ||
:Not so for: 1 with 3; and 2 with 4; and 3 with 5. | :Not so for: 1 with 3; and 2 with 4; and 3 with 5. | ||
| − | |style="text-align:right;"|ולא כן הא' עם הג' וב' עם הד' וג' עם ה' | + | |style="text-align:right;"|ולא כן הא' עם הג' וב' עם הד' וג' עם ה‫' |
|- | |- | ||
| | | | ||
| − | :The aspect of half the celestial circle and the | + | :The aspect of half the celestial circle and the aspect of its quarter is that of its half, which is an aspect of hostility in passivity. |
|style="text-align:right;"|ומבט חצי הגלגל ומבט רביעית בחציו והוא מבט איבה בפעול | |style="text-align:right;"|ומבט חצי הגלגל ומבט רביעית בחציו והוא מבט איבה בפעול | ||
|- | |- | ||
| Line 344: | Line 347: | ||
| | | | ||
::We subtract 4 from 11; 7 remain. We multiply this by 11; the result is 77 and this is the deficit of the square of the greatest. | ::We subtract 4 from 11; 7 remain. We multiply this by 11; the result is 77 and this is the deficit of the square of the greatest. | ||
| − | |style="text-align:right;"|חסרנו ד' מי"א נשארו ז'<br> | + | |style="text-align:right;"|חסרנו ד' מי"א נשארו ז‫'<br> |
כפלנוהו על י"א עלה ע"ז והוא חסרון מרבע היתר | כפלנוהו על י"א עלה ע"ז והוא חסרון מרבע היתר | ||
|- | |- | ||
| Line 353: | Line 356: | ||
| | | | ||
::Such as [the ratio of] 4, 8, 9. | ::Such as [the ratio of] 4, 8, 9. | ||
| − | |style="text-align:right;"|כמו ד' ח' ט' | + | |style="text-align:right;"|כמו ד' ח' ט‫' |
|- | |- | ||
| | | | ||
| Line 365: | Line 368: | ||
| | | | ||
:*Because in the acute-angled triangle there can be no smaller number than four. | :*Because in the acute-angled triangle there can be no smaller number than four. | ||
| − | |style="text-align:right;"|כי החד לא יתכן להיות בו פחות מד' | + | |style="text-align:right;"|כי החד לא יתכן להיות בו פחות מד‫' |
|- | |- | ||
| | | | ||
| Line 432: | Line 435: | ||
| | | | ||
*Hence, the beginning of the squares is the four. | *Hence, the beginning of the squares is the four. | ||
| − | |style="text-align:right;"|על כן תחלת המרבע ד' | + | |style="text-align:right;"|על כן תחלת המרבע ד‫' |
|- | |- | ||
| | | | ||
| Line 461: | Line 464: | ||
| | | | ||
*When you add the one that is like a square to the square of the first even number, it is five. <math>\scriptstyle{\color{blue}{1+2^2=5}}</math> | *When you add the one that is like a square to the square of the first even number, it is five. <math>\scriptstyle{\color{blue}{1+2^2=5}}</math> | ||
| − | |style="text-align:right;"|וכשתחבר האחד שהוא דומה למרובע אל מרובע ראשון הזוגות יהיה ה' | + | |style="text-align:right;"|וכשתחבר האחד שהוא דומה למרובע אל מרובע ראשון הזוגות יהיה ה‫' |
|- | |- | ||
| | | | ||
| Line 481: | Line 484: | ||
| | | | ||
::Such as 3, because [the sum of its square and] the square of its double is, the cube is 27, and its ratio to the sum of the squares is as the ratio of 3 to 5. | ::Such as 3, because [the sum of its square and] the square of its double is, the cube is 27, and its ratio to the sum of the squares is as the ratio of 3 to 5. | ||
| − | |style="text-align:right;"|כמו ג' כי מרובעו כפלו מ"ה והמעוקב כ"ז וערכו אל המרובעים כערך ג' אל ה' | + | |style="text-align:right;"|כמו ג' כי מרובעו כפלו מ"ה והמעוקב כ"ז וערכו אל המרובעים כערך ג' אל ה‫' |
|- | |- | ||
| | | | ||
| Line 526: | Line 529: | ||
| | | | ||
*The six consists of 3, because the boundary of the body is threefold: | *The six consists of 3, because the boundary of the body is threefold: | ||
| − | |style="text-align:right;"|הששה מורכב מן ג' כי גבול הגוף ג' | + | |style="text-align:right;"|הששה מורכב מן ג' כי גבול הגוף ג‫' |
|- | |- | ||
| | | | ||
| Line 575: | Line 578: | ||
| | | | ||
*It resembles the one, because it is followed by a number that consists only of two, and then by 9, that consists of three. | *It resembles the one, because it is followed by a number that consists only of two, and then by 9, that consists of three. | ||
| − | |style="text-align:right;"|והנה ידמה לאחד כי אין אחריו מורכב אך משנים גם אח"כ ט' שהוא מורכב מן ג' | + | |style="text-align:right;"|והנה ידמה לאחד כי אין אחריו מורכב אך משנים גם אח"כ ט' שהוא מורכב מן ג‫' |
|- | |- | ||
| | | | ||
| Line 587: | Line 590: | ||
| | | | ||
*It resembles the 3, because its distance from the analogous is 3, therefore there is a 9 in its the nine squares. | *It resembles the 3, because its distance from the analogous is 3, therefore there is a 9 in its the nine squares. | ||
| − | |style="text-align:right;"|גם ידמה לג' כי מרחקו מהדומה ג' ע"כ במרובעו ט' | + | |style="text-align:right;"|גם ידמה לג' כי מרחקו מהדומה ג' ע"כ במרובעו ט‫' |
|- | |- | ||
| | | | ||
*It resembles the four, because the scales of a square is a square or 7. | *It resembles the four, because the scales of a square is a square or 7. | ||
| − | |style="text-align:right;"|גם ידמה לארבעה כי כל מאזני מרובע מרובע או ז' | + | |style="text-align:right;"|גם ידמה לארבעה כי כל מאזני מרובע מרובע או ז‫' |
|- | |- | ||
| | | | ||
| Line 623: | Line 626: | ||
| | | | ||
:*For the [number of the combinations of] two out of seven is 21, which is the product of 3 that is non-even number by 7. | :*For the [number of the combinations of] two out of seven is 21, which is the product of 3 that is non-even number by 7. | ||
| − | |style="text-align:right;"|כי השנים בחשבון שבעה כ"א שהוא כפל ג' שאינו זוג על ז' | + | |style="text-align:right;"|כי השנים בחשבון שבעה כ"א שהוא כפל ג' שאינו זוג על ז‫' |
|- | |- | ||
| | | | ||
| Line 640: | Line 643: | ||
| | | | ||
*Therefore, the word eight is derived from ''As for Asher, his bread shall be fat'' [Genesis 49,20]. | *Therefore, the word eight is derived from ''As for Asher, his bread shall be fat'' [Genesis 49,20]. | ||
| − | |style="text-align:right;"|על כן שמונה מגזרת ''מאשר שמנה לחמו''<ref>בראשית מט, כ</ref> | + | |style="text-align:right;"|על כן שמונה מגזרת ''מאשר שמנה לחמו''‫<ref>בראשית מט, כ</ref> |
|- | |- | ||
| | | | ||
| Line 660: | Line 663: | ||
| | | | ||
:The scales of 6 are as the scales of 3. | :The scales of 6 are as the scales of 3. | ||
| − | |style="text-align:right;"|ומאזני ו' כמאזני ג' | + | |style="text-align:right;"|ומאזני ו' כמאזני ג‫' |
|- | |- | ||
| | | | ||
:The scales of 4 are as the scales of 5. | :The scales of 4 are as the scales of 5. | ||
| − | |style="text-align:right;"|ומאזני ד' כמאזני ה' | + | |style="text-align:right;"|ומאזני ד' כמאזני ה‫' |
|- | |- | ||
| | | | ||
| Line 681: | Line 684: | ||
| | | | ||
*Therefore, the scale of any number that is multiplied by itself or by another is the 9. | *Therefore, the scale of any number that is multiplied by itself or by another is the 9. | ||
| − | |style="text-align:right;"|על כן מאזני כל מספר הכפול על עצמו או על אחר הם ט' | + | |style="text-align:right;"|על כן מאזני כל מספר הכפול על עצמו או על אחר הם ט‫' |
|- | |- | ||
| | | | ||
| Line 693: | Line 696: | ||
| | | | ||
:*because 9 by 9 is 81: the 1 that is the units is on the left, and 80 that is the tens corresponding to the 8 is on the right. | :*because 9 by 9 is 81: the 1 that is the units is on the left, and 80 that is the tens corresponding to the 8 is on the right. | ||
| − | |style="text-align:right;"|כי ט' על ט' פ"א הנה הא' בשמאל שהוא האחדים ובימין הפ' שהוא העשרות כנגד ח' | + | |style="text-align:right;"|כי ט' על ט' פ"א הנה הא' בשמאל שהוא האחדים ובימין הפ' שהוא העשרות כנגד ח‫' |
|- | |- | ||
| | | | ||
| Line 747: | Line 750: | ||
|- | |- | ||
|} | |} | ||
| + | |||
| + | == Notes == | ||
| + | |||
| + | <div class="mw-collapsible mw-collapsed"><div class="mw-collapsible-content"> | ||
| + | <references /> | ||
| + | </div></div> | ||
== Appendix: Bibliography == | == Appendix: Bibliography == | ||
| Line 761: | Line 770: | ||
:4) Hamburg, Staats- und Universitätsbibliothek, Ms. Levy 113/3 (IMHM: f 1559), ff. 24v-27v (15th century) | :4) Hamburg, Staats- und Universitätsbibliothek, Ms. Levy 113/3 (IMHM: f 1559), ff. 24v-27v (15th century) | ||
:5) London, British Library Add. 27038/6 (IMHM: f 5716), ff. 41v-44v (cat. Margo. 1073, 6) (16th century) | :5) London, British Library Add. 27038/6 (IMHM: f 5716), ff. 41v-44v (cat. Margo. 1073, 6) (16th century) | ||
| − | :6) London, British Library Add. 27153/8 (IMHM: f 5826), ff. 37v-40v (cat. Margo. 1085, 8) (Siena, 1431) | + | :6) London, British Library Add. 27153/8 (IMHM: f 5826), ff. 37v-40v (cat. Margo. 1085, 8) (Siena, 1431)<br> |
| + | :[[https://www.bl.uk/manuscripts/Viewer.aspx?ref=add_ms_27153_fs001r London 27153]] | ||
:7) Moscow, Russian State Library, Ms. Guenzburg 338/6 (IMHM: f 47620), ff. 51r-54v (16th-17th century) | :7) Moscow, Russian State Library, Ms. Guenzburg 338/6 (IMHM: f 47620), ff. 51r-54v (16th-17th century) | ||
| − | :8 | + | :8) München, Bayerische Staatsbibliothek, Cod. hebr. 36/9 (IMHM: f 1166), ff. 136r-137v (Istanbul, 1485) |
| − | + | :9) Napoli, Biblioteca Nazionale Vittorio Emanuele III F 12/33 (IMHM: f 11526), ff. 187r-190r (Benevento, 1492) | |
| − | : | + | :10) New York, Jewish Theological Seminary Ms. 2316/2 (IMHM: f 28569), ff. 1v-5r (15th century) |
| − | : | + | :11) New York, Jewish Theological Seminary Ms. 2321/6 (IMHM: f 28574), ff. 44r-46v (1555) |
| − | : | + | :12) New York, Jewish Theological Seminary Ms. 2349/2 (IMHM: f 28602), ff.?? (16th century) |
| − | : | + | :13) New York, Jewish Theological Seminary Ms. 2497/3 (IMHM: f 28750), ff. 7r-8v (15th century) |
| − | : | + | :14) New York, M. Lehmann 43/1 (IMHM: f 23218), ff. 1-4 (19th century) |
| − | : | + | :15) Oxford, Bodleian Library MS Mich. 238/6 (IMHM: f 22048), ff. 52v-56r (cat. Neub. 1234, 6) (17th century) |
| − | : | + | :16) Oxford, Bodleian Library MS Mich. 400/6 (IMHM: f 19291), ff. 14r-15r (cat. Neub. 2006, 3) (15th century) |
| − | : | + | :17) Oxford, Bodleian Library MS Poc. 209/3 (IMHM: f 22049), ff. 23r-26r (cat. Neub. 1235, 3) (15th century) |
| − | : | + | :18) Paris, Bibliothèque Nationale de France heb. 1085/5 (IMHM: f 14919), ff. 53v-55v (15th-16th century) |
| − | : | + | :19) Roma, Biblioteca Casanatense 3104/3 (IMHM: f 74), ff. 17r-21r (15th-16th century) |
| − | : | + | :20) Vatican, Biblioteca Apostolica ebr. 429/19 (IMHM: f 502), ff. 75r-77v (14th-15th century) |
| − | : | + | :21) Warszaw, Żydowski Instytut Historyczny 150/5 (IMHM: f 30154), ff. 33r-35r (19th century) |
| − | : | + | :22) Wien, Öesterreichische Nationalbibliothek Cod hebr. 152/1 (IMHM: f 1422), ff. 1r-3v (18th-19th century)<br> |
| − | : | ||
| − | |||
| + | <span style=color:blue>The transcript is based mainly on manuscript London 27153</span> | ||
'''<u>Edition</u>''':<br> | '''<u>Edition</u>''':<br> | ||
Latest revision as of 17:19, 8 July 2022
| [1]בשם האחד לבדו יחל אברהם עבדו | |
Contents[hide]One |
|
|
האחד סופר את עצמו ואין אחר סופרו[2] והוא יספור כל מספר[3] |
|
והוא שורש ויסוד ומרובע ומעוקב[4] |
|
והוא דומה לעצם הדבר הנושא כל המקרים[5] |
|
וכל מספר בכחו[6] והוא בכל מספר במעשה[7] |
|
והוא ההוה וכל מספר הוה בעבורו[8] |
|
והוא קדמון לכל וכל מספר מתחדש[9] |
|
והוא סבת כל מספר זוג ושאינו זוג |
|
והוא אינו מספר[10] |
|
ולא ירבה ולא יתחלק והוא סבת כל רבוי וחילוק[11] |
|
ואין לו דמיון גם אין לו הפך ולא שינוי[12] |
|
והוא יעשה בפאה אחת מה שיעשה כל חשבון בשתי פאות[13] |
|
והוא כולל כל כלל וכל חלק כי הוא ראשון[14] ואחרון ואין לו שבר[15] כי אם במחשבת חלק הכלל שיהיה אחד |
|
והוא תחלת כל מספר שאינו זוג המחוברים[16] על סדר המולידים המרובעים[17] |
|
גם הוא תחלת כל זוג שתהיינה הקצוות כפל זה על זה שוות[18] |
|
ואם המספר איננו זוג יהיה הכפל ומרובע האמצעי[19] |
|
ובין מרובע למרובע דמות חלק וחצי חלק[20] |
|
ובעבור שאחד אינו שבור היו השנים חלקים נכונים[21] וכן כל חלק וחלק כשנים[22] |
|
וכל צורה משולשת שהיא תחלת הצורות ועליה תתחלק כל הצורה[23] |
|
ואם היה הצלע האחר ג' אחד לא יתכן להיות המשולש כי [24]אם שוה כולו או שוה השוקים רק חדי המקצוע כי המכונה תהיה אחת |
|
וחשבון עשרה דומה לאחת וככה מאה ואלף על הסדר[25] |
|
וחכמי המספר ברצותם לכפול חשבון על חשבון במערכה אחת או בשתים יחסרו אחד למוסד והאריכו לתת טעם |
|
והעקר כי המערכת השנית שם יחל הדמיון |
|
ובעבור הקל על התלמידים חשבו המערכת הראשונה שהם האחדים והוסיפו השנים על השנים כמספרם על כן הוצרכו לחסר אחת |
|
כי מערכת העשרות באמת אחת והמאות שנים והאלפים שלשה והדבר שוה |
Two |
|
|
השנים תחלת כל מספר |
|
ונקרא כן בעבור שהוא מקבל שינוי מגזרת ודתיהם שונות[26] |
|
ומחברתו כמו כפלו בעצמו |
|
וכל [יש][27] עצם וצורה ולא כן האחד |
|
וכל משולש שתהא הצלע האחת שתים ולא יתכן להיותו כי אם שוה כולו או שוקיו שוות או המקצוע רחב |
|
ודע כי כל משולש מתחלק לשנים חלקים שונים |
|
האחד שוה והוא על שנים דרכים שוה כולו או שתי שוקיו ולעולם יהיו כל המקצועים חדים |
|
והחלק השני תהיינה הצלעות משתנות והוא על שלשה דרכים |
|
האחד שיהיה המקצוע רחב כי שתי המקצועות לעולם חדים |
|
ומרובע הצלע הגדול הוא מוסיף על שני מרובעי הצלעות [הקטנות][28] ככפל אחד חלקי קו הצלע על החלק השני עם התוספת על הצלע עד היותה לנוכח העמוד שהוא מבחוץ |
|
ותחלת כל משולש רחב המקצוע על הסדר בג"ד |
|
והדרך השני להיות המקצוע האחד ישר גם השנים מקצועות יהיו חדים |
|
ומרובעי השנים הקטנות כמרובע היתר שהוא הצלע הגדול |
|
והדרך השלישי יקרא חד[29] המקצוע כי כל מקצועותיו חדים |
Three |
|
|
השלשה הפך האחד כי מחברת אחד אל אחד גדול מכפלו בעצמו וחשבון השנים שוה ומחברת ג' אל ג' קטן מכפלו בעצמו ועל דרך שלשה הוא בכל חשבון |
|
והוא תחלת מספר משולש שוה |
|
ומרובעו כמספר תחלת משולש [בלתי שוה][30] |
|
ומעקבו[31] מספר צלעות השוה שהם במערכת הראשונה |
|
ומספר שלשה תחלת כל מספר שאיננו זוג וכפלו על עצמו גדול ממנו |
|
ואין מספר שיהיה המספרים לפניו כמותו רק הוא לבדו |
|
על כן היה חשבון ששה שהוא מורכב ממנו ישר עם חלקיו |
|
והמורכב מהשלשה תחלת החשבון שחלקיו רבים ממנו |
|
ואם תחברנו עם חלקיו יהיה מספר המחובר חשבון ישר |
|
ודע כי האחד כמו אורך שהוא קו |
|
[והשנים שטח][32] |
|
[והשלשה][33] גוף כי יש לו אֹרך ורֹחב ועומק |
|
ואמרו חכמי התושייה כי הזמן מתחלק על שלשה |
|
הנפש הצומחת והחיה והחכמה שלש |
|
והוא תחלת משלש ישר המקצוע |
Four |
|
|
הארבעה מרובע ראשון נראה |
|
והוא תחלת החשבון זוג הזוג |
|
והוא תחלת החשבון מורכב |
|
כי מערכת הראשונה הם ט' והנה האחד יסוד החשבון ונשארו ארבעה ראשונים והם בגה"ז והארבעה האחרים הם מורכבים |
|
ובחשבון המספרים לפניו הם עשרה שהוא תחלת הכלל הדומה והשורש ד' |
|
ובעבור היות האחדים אל הראשון לאחד שאינו מתחלק על כן הוא להפך |
|
וככה ב' עם ה' וג' עם ו' וד' עם ז' וככה [34]עד אין קץ |
|
על כן המזל הרביעי הפך הראשון כי החֹם והקֹר הם הפועלים והשנים האחרים לח ויבש פעולים |
|
והנה כל רביעי במזלות הפך הראשון בפֹעל ומזלות האש לבדה הפך בפֹעל ובפעול |
|
ועל כן אמרו חכמי המזלות כי מבט רביעית הגלגל שנאה ומבט ששית חצי אהבה |
|
כי השלישי מהמזלות על הראשון בתולדת אחד בפֹעל והפך בפעול על כן אמרו כי הוא חצי אהבה |
|
ומבט שלישית אהבה גמורה כי מבט שלישית הגלגל שהוא החמישי בתולדת הראשון על כן מבט אהבה גמורה בפֹעל ובפעול |
|
וככה א' עם ה' כי שניהם שומרים עצמם |
|
וככה ב' עם ו' כי שניהם זוג הנפרד |
|
וככה ג' עם ז' כי שניהם אינם זוגות ומחלקיהן שוה |
|
ולא כן הא' עם הג' וב' עם הד' וג' עם ה' |
|
ומבט חצי הגלגל ומבט רביעית בחציו והוא מבט איבה בפעול |
|
ואל תתמה כי גם הבית השביעי יורה על העזר בדרך שהזכיר בעל ספר יצירה אמ"ש בזכר ואש"מ בנקבה |
|
והנה הגלגל שהוא אחד יחלקהו האלכסון |
|
גם יתחלק האלכסון והנה מבטו רביעית |
|
ואם תשים נקדה ברביעית יתחלק הגלגל לשלשה חלקים שוים למשלש וחציו ששית הגלגל |
|
ולא ימצא מספר אחר שיחלקהו במחשבה בשברים רבים |
|
והארבעה תחלת משלש משתנה חד המקצוע |
|
ואחריו כל המספר הולך על הסדר בכל דרכיו |
|
והנה בעל הרֹחב הראשון יהיה תוספת היתר על השתים כמספר האמצעית והמקצוע החד יחסר מהיתר כמספר האמצעית |
|
והנה אתן לך כלל אם יהיו שלשה מספרים סמוכים על הסדר מארבעה ולמעלה ותרצה לדעת כמה יתרון השתים על היתר חסר לעולם מהאמצעית ארבעה וכפֹל הנשאר על האמצעית |
|
וככה יהיה דמיון יו"ד י"א י"ב |
|
חסרנו ד' מי"א נשארו ז' כפלנוהו על י"א עלה ע"ז והוא חסרון מרבע היתר |
|
ודע כי יש מקצוע רחב מאד ויש קרוב מישר |
|
כמו ד' ח' ט' |
|
וככה יש חד שהוא חצי ישר או שלישיתו או פחות |
|
ואל תחשֹׁב שתוכל לעשות משלש מכל מספר שתרצה |
|
כי החד לא יתכן להיות בו פחות מד' |
|
ולא משלש שתהיה הצלע גדולה מן השתים |
|
ולא לעשות ישר אם היו מספרי המקצועות רחוקים |
|
רק על מרכבת אחת כמו ג' ד' ה' או במֹרכב ממנה |
|
או שתהיינה הצלעות הקטנות סמוכות כמו כ' כ"א כ"ט |
|
או הגדולות סמוכות כמו ה' י"ב י"ג |
|
ויש רחב המקצוע רב כמו י' י"ז כ"ו |
|
ויש מעט כמו י' כ"ג כ"ו |
|
והנה י' כ"ד כ"ו |
|
רק י' כ"ה כ"ו חד מעט |
|
על כן לא נוכל לעשות גם רחב גם חד שתהיינה הצלעות רחוקות |
| I shall give you a rule: | והנה אתן לך כלל |
|
דע כי מדרך החשבון היו המשלשים משֻני צלעות שהם במערכה ראשונה ע"ט והנה אין נכונים מהם רק ל"ג |
|
גם המשלשים בעלי השוקים השוות אין כלם נכונים |
|
והנה חשבון ד' תחלת חשבון שני |
|
על כן כל מספר זוג לעולם יהיה מספר שני שהוא מֻרכב חוץ מחשבון שתים בעבור כח האחד כי הוא ראש מעשיו |
|
והנה כל חשבון ערוך על אחד לא יוסיף כי הוא עצם כל חשבון |
|
על כן תחלת המרבע ד' |
|
וכל מרבע כפול על מרבע מרבע |
|
גם מחֻלק על מרבע מרבע |
|
וערך מרבע אל מרבע מרבע |
|
על כן מדות כל החכמים במרבעים |
Five |
|
|
החמשה חשבון עגול והיה כן יען כי סוף המערכת הראשונה ט' על כן הוא מתגלגל על עצמו כאשר אפרש |
|
וכשתחבר האחד שהוא דומה למרובע אל מרובע ראשון הזוגות יהיה ה' |
|
ואם תחברנו אל מרובע ראשון מחשבון שאינו זוג יהיה כפלו |
|
וכשתחבר מרובעו אל מרובע כפלו יהיה המחובר כמספר מעוקבו |
|
ואם כפלת זה המספר יהיה מרובעו עם מרובע כפלו חצי מעוקבו |
|
ועוד אם בקשת לדעת ערך מרובע איזה מספר שתרצה ומרובע כפלו אל מעוקבו לעולם יהיה כערך המספר אל ה' אם הוא פחות ממנו או ערך ה' אליו אם הוא גדול ממנו |
|
כמו ג' כי מרובעו כפלו מ"ה והמעוקב כ"ז וערכו אל המרובעים כערך ג' אל ה' |
|
וערך ו' מרובעו וכפלו ק"פ והמעוקב רי"ו והנה המספר ה' ששיות המעוקב |
|
ועל זה הדרך הכל |
|
וזה המספר מחובר מתחלת זוג ומתחלת שאינו זוג |
|
ויש אומרים כי חמש הרגשות כנגד זה המספר |
|
ואמרו כי מראה העין מתולדות הכוכבים ע"כ תראה העין בלא זמן |
|
והריח מתולדות האש כי הוא כמי הדם |
|
והטעם מתולדות המים והעקר הליחה |
|
והשמע מתולדות הרוח |
|
והמשוש מתולדות הארץ |
Six |
|
|
הששה מורכב מן ג' כי גבול הגוף ג' |
|
מן האורך יצא פנים ואחור |
|
מן הרוחב ימין ושמאל |
|
ומן העומק מעלה ומטה |
|
והנה מן ג' פיאות נכבדות הפנים |
|
כי תנועת כל החיים לפנים גם לימין כי כן תנועת הגלגל הגדול גם מעלה כי כן תנועת כל צומח למעלה |
|
וכבר הזכרתי שהוא ישר |
Seven |
|
|
השבעה מחובר מתחלת מספר שאיננו זוג עם שני לזוג |
|
וככה הוא מחובר מתחלת זוג עם שני לאשר איננו זוג |
|
ובעבור זה קראוהו חכמי התושיה חשבון שלם |
|
ואמר עליו בעל ספר יצירה כי הוא מכוון באמצע והנה הוא סוד האחד שאינו גוף עם הגוף שיש לו ו' פיאות |
|
והנה ידמה לאחד כי אין אחריו מורכב אך משנים גם אח"כ ט' שהוא מורכב מן ג' |
|
והנה הוא כתחלת חשבון |
|
גם ידמה לשנים כי מאזני מרובעיהם שוים |
|
גם ידמה לג' כי מרחקו מהדומה ג' ע"כ במרובעו ט' |
|
גם ידמה לארבעה כי כל מאזני מרובע מרובע או ז' |
|
גם דומה לחמשה כי המחובר מרובע אחד ומרובע תחלת הזוג ואשר איננו זוג כפול ז' שהוא י"ד |
|
ויגיע עד מרובע ז' ק"מ כדמות י"ד |
|
גם ידמה לששה כי אל ז' המחובר שלפניו חשבון ישר עם חלקיו בשוה |
|
ועוד כי כחשבון שלושה המשרתים ויש לו סוד |
|
כי אם יהיו ד' לא יהיו שנים במספר |
|
וככה אם יהיו ה' לא יהיו השנים כן עשרה ואם הוא מחמשה הנה לא יהיה זוג הפך תולדותו |
|
וככה ששה וכל מספר אחרי שבעה |
|
כי השנים בחשבון שבעה כ"א שהוא כפל ג' שאינו זוג על ז' |
|
וככה הם הרבעיים |
Eight |
|
|
השמונה ידוע כי האורך בין שתי נקודות והנה המרחב ד' נקודות והגוף ח' והוא העובי |
|
על כן שמונה מגזרת מאשר שמנה לחמו[35] |
|
גם הוא חשבון מעוקב |
|
ובעבור שהם ח' גלגלים גדולים שהם העגולות |
|
הנה מאזני ח' כמו אחד |
|
ומאזני ז' כמו שנים |
|
ומאזני ו' כמאזני ג' |
|
ומאזני ד' כמאזני ה' |
|
וכנגד הח' האש והרוח והמים והעפר כי לכל אחד ואחד ב' תולדות כי הם מורכבים מארבע יסודות |
Nine |
|
|
התשעה סוף המערכת הראשונה |
|
על כן מאזני כל מספר הכפול על עצמו או על אחר הם ט' |
|
והוא תחלת מרובע מספר שאינו זוג |
|
ואם תעשה עגול ותכתוב בו הט' מספרים הנה ט' מתגלגל על כל המספרים שהם לפניו |
|
כי ט' על ט' פ"א הנה הא' בשמאל שהוא האחדים ובימין הפ' שהוא העשרות כנגד ח' |
|
ואח"כ מהשמאל ט' על ח' ע"ב ב' בשמאל וז' בימין בעשרות |
|
ואח"כ מהשמאל ג' ובימין ו' שהוא ט' על ז' ס"ג |
|
ואח"כ מהשמאל ד' באחדים ובימין ה' בעשרות והוא כפל ו' על ט' נ"ד |
|
ואחר כך בשמאל ה' ושב מספר מ' כנגד הד' שיהיו אחדים כי תהפוך הגלגל ושבו החמשים חמשה |
|
גם הט' על ד' עלו ל"ו והנה האחדים בימין והעשרות בשמאל |
|
ג"כ כ"ז |
|
גם י"ח |
|
ועוד נחשוב כי שתים עגולות הם כנגד העליונות האחת מזרחית והשנית מערבית ונאמר כי גם הט' בראש התלי מהגלגל וזאת הולכת לצד אחד והאחרת לצד אחר |
|
והנה מרובע ט' יש בו א' וככה כלם ע"כ היה חשבון ה' באמצע כאלו היא בזנב התלי ע"כ הוא חשבון עגול |
| I will show you a method for the squares: | והנה אראה לך דרך במרובעים |
| We know that the hundreds are similar to the units and the squares are also similar to those that are in the first rank. | ידענו כי המאות דומות לאחדים ושבו מרובעים דומים לאשר הם במערכת הראשונה |
|
והנה בקשנו לדעת כמה מרובע י"ט |
|
כי הקרוב אליו ת' וחשבונו לפניו והנה נחסר אחד מהשלמת העשרים והנה נכפלנו ונחסרנו אחורנית יעלה המספר ש"ס ונוסיף מרובע הא' שחסרנו והנה עלה הכל שס"א וזה מרובע י"ט |
Notes
[Expand]
Appendix: Bibliography
Abraham ben Meir Ibn ‛Ezra
b. 1089, Tudela, Spain – d. 1167
Sefer ha-ʼEḥad (The Book of One)
Prior to 1148, possibly in Béziers
Manuscripts:
- 1) Berlin, Staatsbibliothek (Preußischer Kulturbesitz) Or. Oct. 244/12 (IMHM: f 1996), ff. 57v-59v (15th-16th century)
- 2) Budapest, Magyar Tudományos Akadámia, MS. Kaufmann A 271/6 (IMHM: fiche 76), ff. 417-424 (15th century)
- 3) Cambridge, University Library Add. 1215, 3/3 (IMHM: f 17078), ff. 25v-28v (1532)
- 4) Hamburg, Staats- und Universitätsbibliothek, Ms. Levy 113/3 (IMHM: f 1559), ff. 24v-27v (15th century)
- 5) London, British Library Add. 27038/6 (IMHM: f 5716), ff. 41v-44v (cat. Margo. 1073, 6) (16th century)
- 6) London, British Library Add. 27153/8 (IMHM: f 5826), ff. 37v-40v (cat. Margo. 1085, 8) (Siena, 1431)
- [London 27153]
- 7) Moscow, Russian State Library, Ms. Guenzburg 338/6 (IMHM: f 47620), ff. 51r-54v (16th-17th century)
- 8) München, Bayerische Staatsbibliothek, Cod. hebr. 36/9 (IMHM: f 1166), ff. 136r-137v (Istanbul, 1485)
- 9) Napoli, Biblioteca Nazionale Vittorio Emanuele III F 12/33 (IMHM: f 11526), ff. 187r-190r (Benevento, 1492)
- 10) New York, Jewish Theological Seminary Ms. 2316/2 (IMHM: f 28569), ff. 1v-5r (15th century)
- 11) New York, Jewish Theological Seminary Ms. 2321/6 (IMHM: f 28574), ff. 44r-46v (1555)
- 12) New York, Jewish Theological Seminary Ms. 2349/2 (IMHM: f 28602), ff.?? (16th century)
- 13) New York, Jewish Theological Seminary Ms. 2497/3 (IMHM: f 28750), ff. 7r-8v (15th century)
- 14) New York, M. Lehmann 43/1 (IMHM: f 23218), ff. 1-4 (19th century)
- 15) Oxford, Bodleian Library MS Mich. 238/6 (IMHM: f 22048), ff. 52v-56r (cat. Neub. 1234, 6) (17th century)
- 16) Oxford, Bodleian Library MS Mich. 400/6 (IMHM: f 19291), ff. 14r-15r (cat. Neub. 2006, 3) (15th century)
- 17) Oxford, Bodleian Library MS Poc. 209/3 (IMHM: f 22049), ff. 23r-26r (cat. Neub. 1235, 3) (15th century)
- 18) Paris, Bibliothèque Nationale de France heb. 1085/5 (IMHM: f 14919), ff. 53v-55v (15th-16th century)
- 19) Roma, Biblioteca Casanatense 3104/3 (IMHM: f 74), ff. 17r-21r (15th-16th century)
- 20) Vatican, Biblioteca Apostolica ebr. 429/19 (IMHM: f 502), ff. 75r-77v (14th-15th century)
- 21) Warszaw, Żydowski Instytut Historyczny 150/5 (IMHM: f 30154), ff. 33r-35r (19th century)
- 22) Wien, Öesterreichische Nationalbibliothek Cod hebr. 152/1 (IMHM: f 1422), ff. 1r-3v (18th-19th century)
The transcript is based mainly on manuscript London 27153
Edition:
- Ibn ‛Ezra, Abraham. Sefer ha-’Eḥad. In: Yalqut Avraham Ibn ‛Ezra, ed. Israel Levin. New York, Tel Aviv: Israel Matz Hebrew Classics Ltd. 1985, pp. 399-414.
- Ibn Ezra, Abraham. Sepher ha-Echad: liber de novem numeris cardinalibus. Ed. Simchae Pinsker and Michael Abba Goldhardt. Odessa: 1867.
Bibliography:
- Langermann, Y. Tzvi. 2001. Studies in Medieval Hebrew Pythagoreanism: Translations and Notes to Nicomachus; Arithmological Texts, Micrologus IX, pp. 219–236.
- Sela, Shlomo, and Gad Freudenthal. 2006. Abraham Ibn Ezra’s scholarly writings: a chronological listing, Aleph 6, pp. 13-55.
Commentary on Sefer ha-ʼEḥad
Mordecai ben Eliezer Comṭino
Constantinople & Adrianople c. 1402-1482
Manuscripts:
- 1) Moscow, Russian State Library, Ms. Guenzburg 469/5 (IMHM: f 43040), ff. 184r-211r (16th century)
- 2) New York, Jewish Theological Seminary Ms. 2577/1 (IMHM: f 28830), ff. 1r-31v (19th century)
- 3) New York, M. Lehmann 43/2 (IMHM: f 23218), ff. 6-24 (19th century)
- 4) Paris, Bibliothèque Nationale de France heb. 681/3 (IMHM: f 11559), ff. 79v-101r (15th century)
- 5) Parma, Biblioteca Palatina Cod. Parm. 2446/3 (IMHM: f 13450), ff. [72r]-[89v] (15th century)
- 6) St. Petersburg, Inst. of Oriental Studies of the Russian Academy A 221/3 (IMHM: f 69628), ff. :24v-42v (1472)
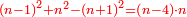
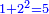
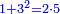
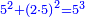
![\scriptstyle{\color{blue}{\left(2\sdot5\right)^2+\left[2\sdot\left(2\sdot5\right)\right]^2=\frac{1}{2}\sdot\left(2\sdot5\right)^3}}](/mediawiki/images/math/c/c/8/cc8d772d9367fc32e176a5ca9156e880.png)