Difference between revisions of "האריתמטיקה של ניקומכוס"
(→Absolute quantity) |
(→Introduction) |
||
| Line 1: | Line 1: | ||
| + | {{#annotpage: author="Nicomachus of Gerasa", translator="Qalonymos ben Qalonymos", country="South of France", time="1286-1329", peshat_author="00000008", peshat_title="00002022"}} | ||
__TOC__ | __TOC__ | ||
<br> | <br> | ||
| Line 7: | Line 8: | ||
== Book One == | == Book One == | ||
| − | !style="text-align:right;"|המאמר הראשון מספר הארתמאיטי | + | !style="width:45%;text-align:right;"|<big>המאמר הראשון מספר הארתמאיטי</big> |
|- | |- | ||
| | | | ||
| − | === Prologue | + | === <span style=color:green>[Prologue by al-Kindī's Student]</span> === |
| | | | ||
| + | |- | ||
| + | |May God fulfill your wishes, carry out your wishes and increase His grace and His pleasantness upon you. | ||
| + | |style="text-align:right;"|<big>ימלא</big> הבורא משאלותיך ויפיק זממיך ויוסיף חסדו ונעמו אצלך | ||
| + | |- | ||
| + | |<span style=color:green>[The history of the successive translations of the text:]</span> | ||
| + | | | ||
| + | |- | ||
| + | |I have understood - may God perpetuate your glory - what you have mentioned: that you had studied the famous arithmetic book that is among us, | ||
| + | |style="text-align:right;"|הבינותי יתמיד האל כבודך מה שזכרת כי עיינת בספר הארתמאיטיקא הידוע אשר בינינו | ||
| + | |- | ||
| + | | | ||
| + | *which has been written and composed by the Pythagorean '''Nicomachus al-Gehrasīnī''' [= of Gerasa], | ||
| + | |style="text-align:right;"|אשר הניחו וחברו ניקומאכוש אלגהר שיני הפיתאגורי | ||
| + | |- | ||
| + | | | ||
| + | *in a version, according to which we have revised this book, on the authority of our noble master, the Revised by '''Ya‛qūb b. Isḥaq al-Ṣabbāḥ al-Kindī''', | ||
| + | |style="text-align:right;"|בנסחא אשר תקננו זה הספר ממנה על דעת מלמדנו המעולה יעקב בן אסחק אלצבאה אלכנדי | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *who doubted the thoughts of the Nestorian '''Ḥabīb Ibn Bahrīz''', who translated it from Syrian into Arabic for '''Ṭāhir b. al-Ḥusayn''' the ambidextrous. |
| + | |style="text-align:right;"|משבש מחשבות חביב בן בהריז אלנסטורי אשר תרגמו מהסריאני אל הערבי לבעל שני הימינים טאהר בן אלחסין | ||
| + | |- | ||
| + | |You have wished to revise it in the first section, from the place of the numbers and onwards, for it was indeed revised from this place, since it has already been preceded by that which in the science of logic could help him to understand the previous introduction, before the mentioning of the number, and this introduction did not reach you and you did not know its purpose nor what its author has mentioned in it. | ||
| + | |style="text-align:right;"|ואתה ראית לתקנו במאמר הראשון ממקום המספרים והלאה לפי שהוא אמנם תוקן מזה המקום לפי שכבר קדם לו בחכמת ההגיון מה שיעזרהו להבין פתיחתו הקודמת לפני זכירת המספר ושזאת הפתיחה לא נפלה אליך ולא ידעת כוונתה ולא מה זכר בה מניחה | ||
| + | |- | ||
| + | |What you have asked for in this respect, is explained to you, as I explained it in our other explanations on the book. | ||
| + | |style="text-align:right;"|ואשר שאלת מזה הנו מפורש לך כמו שפירשתי בשאר פירושינו הנשארים בספר | ||
| + | |- | ||
| + | |We have summarized his remarks in a concise discussion, without lengthiness or repetitions. | ||
| + | |style="text-align:right;"|וקצרינו לעניניו במאמר קצר מבלתי אריכות והכפלה | ||
| + | |- | ||
| + | |You think it a precious benefit and a wonderful guidance. | ||
| + | |style="text-align:right;"|ואתה חושב שבזה תועלת יקרה והערה נפלאה | ||
| + | |- | ||
| + | |But I inform you, may God grant you honor, that many times I perhaps wished to describe something in detail and at length, in order to reach the intention of the author of the book. | ||
| + | |style="text-align:right;"|ואני מודיעך יכבדך האל אולי ארצה הרבה פעמים לתאר דבר בהפלגה ואריכות כדי להגיע בו אל כוונת מניח הספר | ||
| + | |- | ||
| + | |Then, I came across a concise statement I heard from our master, '''Abū Yusūf''', who already succeeded in explaining what you had requested to explain and in interpreting what you had intended to interpret, in spite of his brevity, as a long speech would not have achieved and a detailed description would not have surpassed. | ||
| + | |style="text-align:right;"|ויזדמן לי מאמר קצר שמעתיו ממלמדנו אבו יוסף לבאר מה ששאלת לבארו ולפרש מה שכוונת לפרשו כבר הגיע ממנו עם קצורו כאשר לא יגיעהו מאמר מאריך ולא יעברהו תאר מפליג | ||
| + | |- | ||
| + | |So, I have abandoned what I wanted to reveal through long words, in favor of his concise speech, and because of this, I will refrain from embellishing the book and decorating it more. | ||
| + | |style="text-align:right;"|ואעזוב מה שראיתי לגלותו בדברים מרובים בעבור מאמרו הקצר ובעבור זה אמנע אני מליפות הספר ולקשטו ביותר | ||
| + | |- | ||
| + | |More than once I have heard our master saying that the philosophy of these two men, i.e. '''Ptolemy''' and '''Nicomachus''', is best clarified in the introduction to their works - Ptolemy in his introduction to the '''Almagest''' and Nicomachus in the introduction to the arithmetic book - for the introductions of these two works stimulate elevated regions of philosophy and they are of valuable virtue in knowledge. | ||
| + | |style="text-align:right;"|וממה ששמעתיו ממלמדנו יותר מפעם אחת כשיאמר שהיותר ראוי ממה שתתבאר בו פילוסופית שני אלו האנשים ר"ל בטלמיוס וניקומאכוש הוא בפתיחת נושאיהם אם בטלמיוס בפתיחתו לספר המגסטי ואם ניקומאכוש בפתיחת ספר הארתמאיטיקא כי פתיחות שני אלו הספרים מעוררות על מקומות עליונים בפילוסופייא ומדרגתם יקרה בידיעה | ||
| + | |- | ||
| + | |Your thought concerning this was not erroneous. Indeed, I thought, by God, that it is true, because the considerable scope of the introduction of this book and the virtue of the quantity of its benefit and goodness are truly comprehended by you. | ||
| + | |style="text-align:right;"|ולא כזבה מחשבתך בזה ואמנם אחשוב אותה חי השם שהיא אמתית למה שהתאמת אצלך גודל מעלת פתיחת זה הספר ומעלת שעור תועלתו וטובו | ||
| + | |- | ||
| + | |In answer to your request, may God glorify you, drawn to appease your mind with anything I can avail for you. | ||
| + | |style="text-align:right;"|ואני השיבותיך יפארך השם למה ששאלת נמשך להפיס דעתך בכל מה שאוכל להועילך | ||
| + | |- | ||
| + | |I wrote to you the introduction of this book in my own work, here, with all that can be explained of it, completing the sufficient speech and omitting any repetition and redundancy. | ||
| + | |style="text-align:right;"|וכתבתי אליך פתיחת זה הספר בספרי זה וכל מה שאפשר לבארו ממנו בהשלמת המאמר המספיק ולהשליך ההכפלה והמותר | ||
| + | |- | ||
| + | |I explained its terms and removed from it its obscurity and difficulty. | ||
| + | |style="text-align:right;"|ואפרש בו מלותיו והסירותי ממנו עמקו וקשיו | ||
| + | |- | ||
| + | |In doing so, I made it more accessible to you than the words of the translator, so that there will be no difference between the meanings. | ||
| + | |style="text-align:right;"|וכבר עשיתי זה והקרבתיו לך ממלת המעתיק אותו מבלתי שיהיה בין הענינים חלוף | ||
| + | |- | ||
| + | |Indeed, what is of a high virtue is far from the custom. | ||
| + | |style="text-align:right;"|ואמנם מה שהיה עליון במדרגה רחוק מהמנהג | ||
| + | |- | ||
| + | |I will not depart from the order of the author of this book. | ||
| + | |style="text-align:right;"|הנני לא אסור מלספר סדר מניח הספר | ||
| + | |- | ||
| + | |But I append to each chapter in this description a discussion attached to that chapter that states the true meaning of the thesis. | ||
| + | |style="text-align:right;"|אבל אני ממשיך לכל פרק שיהיה בזה התאר מאמר סמוך לאותו הפרק מגיד אמתת הדרוש | ||
| + | |- | ||
| + | |I shall not pass beyond the opinion of our master, '''Abū Yusūf''', concerning any thing, to which I will refer. | ||
| + | |style="text-align:right;"|ולא אעבור בדבר ממה שאביא בו דעת מלמדנו אבו יוסף | ||
| + | |- | ||
| + | |Now it is the time to begin with what you asked for. | ||
| + | |style="text-align:right;"|ובכאן עת להתחיל במה ששאלת | ||
| + | |- | ||
| + | |May you succeed in that. | ||
| + | |style="text-align:right;"|והיה בו מצליח | ||
| + | |- | ||
| + | |May God, the exalted, lead you to what will save your soul. | ||
| + | |style="text-align:right;"|יישירך השם יתע' למה שתושע בו נפשך | ||
|- | |- | ||
| | | | ||
| + | |||
=== Introduction === | === Introduction === | ||
| | | | ||
| + | |- | ||
| + | |'''Nicomachus''' '''said''': The first among the ancients who scrutinized the science and deepened in it - the first of them was Pythagoras - defined philosophy by saying that philosophy is the the love of science, as its name indicates this, for its translation is "the love of science". | ||
| + | |style="text-align:right;"|<big>אמר ניקומאכוש</big> שהקדמונים הראשונים אשר דקדקו בחכמה והעמיקו בה והיה הראשון להם פיתאגורש גדרו הפילוסופייא כשאמרו שהפילוסופיאה אהבת החכמה כמו ששמה מורה על זה ממנה כי העתקתו אהבת החכמה | ||
| + | |- | ||
| + | |By saying "as its name indicates this", he meant that the term philosophy is composed of two words "filo" and "sofia" - "filo" is loving and "sofia" is science - so the term is composed from "loving science". | ||
| + | |style="text-align:right;"|ירצה באומרו שמה מורה על זה ממנה לפי ששם הפילוסופיאה מורכב משתי מלות פילו וסופיה ופילו אוהב וסופיה חכמה הנה שמה מורכב מאוהב החכמה | ||
| + | |- | ||
| + | |'''He''' [= Nicomachus] '''said''': Before Pythagoras, whoever was skilled in any craft or medicine was called "wise", in a general term, without investigating the principle by which something should be named by the term "science" | ||
| + | |style="text-align:right;"|<big>אמר</big> וכבר היה אשר היו קודם פיתאגוריש וקראו כל מי שהיה פקח בדבר מהמלאכות או הרפואה חכם בשם משולח מבלתי שיחקרו על השורש אשר בו ראוי להקרא בשם החכמה | ||
| + | |- | ||
| + | |'''<span style=color:blue>Pythagoras</span>''', reserving this name to its meaning and to the root from which it is derived, has called "science" specifically - excluding the sciences that derived from it - the true knowledge of permanent things, i.e. the species and genera. | ||
| + | |style="text-align:right;"|אמנם ''{{#annot:Pythagoras|509|wEI0}}פיתאגוריש{{#annotend:wEI0}}'' למה שייחד זה השם לעיניינו והשרש אשר ממנו נגזר קרא אמתת הידיעה בדברים המתמידים ר"ל המינים והסוגים החכמה בפרט מבלתי מה שיצא מזה מן החכמות | ||
| + | |- | ||
| + | |'''He''' [= Nicomachus] '''said''': it is appropriate to call the science "sofia" and the love of it "philosophy", i.e. the love of science. | ||
| + | |style="text-align:right;"|<big>אמר</big> והראוי מה שיקרא החכמה סופיה והאהבה לה הפילוסופיה ר"ל אהבת החכמה | ||
| + | |- | ||
| + | |'''He said''': The statement about its definition is the more accurate and it should be accepted, rather than [the one of] those who defined it by a different definition, since it was already shown that its definition is derived from its name. | ||
| + | |style="text-align:right;"|<big>אמר</big> הנה יותר ראשון שיצדק המאמר בגדרה ויותר ראוי בשנקבל ממנו בלתי מי שגדרה בזולת זה הגדר אחרי שכבר הורנו על שגדרה נגזר משמה | ||
| + | |- | ||
| + | |'''Abū Yusūf''' '''said''': The ancients defined philosophy by several definitions | ||
| + | :'''<span style=color:green>[A list of definitions of philosophy given by the ancients:]</span>''' | ||
| + | |style="text-align:right;"|<big>אמר אבו יוסף</big> כי הקדמונים גדרו הפילוסופיה במספר גדרים | ||
| + | |- | ||
| + | | | ||
| + | *Derivation of its name, as loving science, since 'filosof' is composed of filo, which is loving, and sofia, which is the science. | ||
| + | |style="text-align:right;"|אם מגזרת שמה כמו אוהב החכמה לפי שפילוסוף הוא מורכב מפילו והוא אוהב וסופיה והוא החכמה | ||
| + | |- | ||
| + | | | ||
| + | *It has also been defined from its effect: it has been said that philosophy is the imitation of the Creator, the exalted, according to the human capacity, meaning that man must be of perfect virtue. | ||
| + | |style="text-align:right;"|ואם שגדרוה מפעולתה ואמרו שהפילוסופיאה ההתדמות בבורא ית' כפי יכולת האדם ירצה בזה שיהיה האדם שלם בחשיבות | ||
| + | |- | ||
| + | | | ||
| + | *It has been further defined from its effect: it has been said that it is the attention paid to death. The death is twofold: natural death, which is when the soul leaves the usage of the body; and a voluntary death, which is the abolition of desires by the sword of the virtuous choices - this is the death aimed for here, for the abolition of desires is the path leading to the excellence. Because of this many of the most eminent philosophers have said that pleasure is necessarily bad. For, when the soul engages in two activities - one of them is sensual and the other of the intellectual - and what is called "pleasure" is what happens when it indulges in the sensual pleasures - the soul then ceases to engage in the intellect. | ||
| + | |style="text-align:right;"|וגדרוה עוד מצד פעולתה ואמרו שהיא ההשגחה במות<br> | ||
| + | והמות אצלם שתי מיתות<br> | ||
| + | מות טבעי והוא עזיבת הנפש שמוש הגוף<br> | ||
| + | ומות רצוניי והוא הריגת התאות בחרב הבחירות החשובות וזהו המות אשר בו כוונו הנה לפי שבמיתת התאוות הדרך להגיע אל החשיבות<br> | ||
| + | ולזה אמרו רבים מחשובי הפילוסופים שהתענוג רע בהכרח שכאשר יהיו לנפש שני עסקים אחד מהם חושיי והאחר שכליי והיה מה שיקרא תענוג הוא מה שיקרה בהרגשה עם העסק בתענוגיה החושיים תעזוב הנפש עם זה העסק בשכל | ||
| + | |- | ||
| + | | | ||
| + | *Philosophy has been also defined by its cause: saying that philosophy is the art of the arts and the science of the sciences. | ||
| + | |style="text-align:right;"|וגדרו גם כן הפילוסופיא מצד העלה כשאמרו הפילוסופיא היא מלאכת המלאכות וחכמת החכמות | ||
| + | |- | ||
| + | | | ||
| + | *Philosophy has been also defined by saying that it is the knowledge that man has of himself. | ||
| + | |style="text-align:right;"|וגדרו גם כן הפילוסופיה כשאמרו כי היא ידיעת האדם עצמו | ||
| + | |- | ||
| + | | | ||
| + | :This saying is very noble, very profound. | ||
| + | |style="text-align:right;"|וזה מאמר נכבד ורחוק מהעול מאד | ||
| + | |- | ||
| + | | | ||
| + | :As an example, it is said that since things are either bodies or non-bodies - and the bodies are substances, while the non-bodies are either substances or accidents - but, man is endowed with a soul, a body and an accident - and the soul is a substance, non-body - thus, when the man knows himself, he knows the body with its accidents, the first accident, and the substance that is a non-body; and when he knows all of this together, he knows all. This is the cause for which the wise men have called the man a microcosm. | ||
| + | |style="text-align:right;"|ודרך משל אומר שהדברים אחר שהיו גשמים ולא גשמים והיו הגשמים עצמים והיו לא גשמים אם עצמים ואם מקרים והיה האדם הוא בעל הנפש והגשם והמקרה והיתה הנפש עצם לא גשם הנה כשידע האדם עצמו ידע הגשם במקריו והמקרה הראשון והעצם אשר הוא לא גשם וכאשר ידע זה יחד הנה כבר ידע הכל ולזאת העלה קראו החכמים האדם עולם קטן | ||
| + | |- | ||
| + | |[Al-Kindī] defines philosophy as the eternal universal things - their essences, their beings, their causes, and their whys, according to the human comprehension capacity. | ||
| + | |style="text-align:right;"|ואמנם מה שנגדור בו אנחנו הפילוסופיה הוא שהפילוסופיה היא ידיעת הדברי' הנצחיים הכוללים ישתויותיהם ומהיותיהם ועלותיהם ולמויותיהם כפי יכולת האדם להשיגו | ||
| + | |- | ||
| + | |The words of Abū Yusūf are completed. | ||
| + | |style="text-align:right;"|שלמו דברי אבו יוסף | ||
| + | |- | ||
| + | |'''The author of this book''' [= Nicomachus] '''said''': he [Pythagoras] further defined science by saying that science is the veracious knowledge of permanent things. | ||
| + | |style="text-align:right;"|<big>אמר מניח הספר</big> ועוד שהוא גדר החכמה בשאמר שהחכמה אמיתת הידיעה בדברים התמידים | ||
| + | |- | ||
| + | |He has also defined knowledge by saying that knowledge is the apprehension of the purpose of things that are ascribed to knowledge, i.e. those that are permanent, whose existence does not change, whose being does not change, and who never depart from their quality. These are the primary natural species and genera, which - when the individuals are associated and described by them - deserve the name of existing, since their species give them names and definitions. | ||
| + | |style="text-align:right;"|עוד גדר הידיעה כשאמר שהידיעה היא השגת תכלית הדברים המיוחסים לידיעה ר"ל אותם התמידיים אשר לא יעתקו מעניין מציאותם ולא ישיגם שינוי בישותם ולא יותרו מתכונתם והם המינים והסוגים הראשונים בטבע אשר בהשתתפות האישים להם וכשהם מתוארים בם היו ראויים לשם הנמצא אחר שיתנו מיניהם שמותיהם וגדריהם | ||
| + | |- | ||
| + | |The bodily perceptible individuals are in perpetual disintegration and in continuous change. They are similar, however, by nature and property, to the first property of the primeval matter from which they were formed. By property, the intention is to the element, because the whole element was a single sphere, which has been transformed and modified. | ||
| + | |style="text-align:right;"|ואמנם האישים המוחשים הגשמיים הנה הם בהתוך מתמיד ובשנוי מדובק והנה עם זה מתדמי הטבע והסגולה אל הסגולה הראשונה מן ההיולי אשר בו התוו ורצוני בסגלה היסוד כי היסוד כלו היה גלגל אחד השתנה והותר | ||
| + | |- | ||
| + | |'''Abū Yusūf''' '''said''': He means that the exalted Creator has made certain qualities as certain causes. | ||
| + | |style="text-align:right;"|<big>אמר אבו יוסף</big> ירצה בזה שהבורא ית' שם קצת תכונה לקצת עלות | ||
| + | |- | ||
| + | |As an example, the sight, which he made as the cause of visible colors, and the hearing, which he made as the cause of the existence of the audibles. | ||
| + | |style="text-align:right;"|ודרך משל אומר כראות אשר שמו עלה לנראים מהגוונים והשמע אשר שמו עלה למציאות הנשמעים | ||
| + | |- | ||
| + | |Likewise, he has made the faculty, which is called the nature, as the cause of the movement of the moving bodies that are resting after the movement. | ||
| + | |style="text-align:right;"|וכן שם הכח אשר יקרא הטבע עלה לתנועות המתנועעים הנחים אחר התנועה | ||
| + | |- | ||
| + | |The moving bodies are the bodies, and because of this the bodies are called nature, since those that receive their movement and their rest by nature are therefore always deficient and changing. | ||
| + | |style="text-align:right;"|והמתנועעים הם הגשמים ולזה יקראו הגשמים טבע לפי שאשר יקבלו מהתנועה והמנוחה אמנם יקבלוהו מן הטבע הנה הם בזה בחסרון לעולם ובתמורה | ||
| + | |- | ||
| + | |Also the perceptible bodies, i.e. the individuals, are always affected by shifting and change. | ||
| + | |style="text-align:right;"|וכן הגשמים המוחשים ר"ל האישים יקרה להם ההעתק והתמורה תמיד | ||
| + | |- | ||
| + | |Those of them that exist are affected by change in substance and in nature. | ||
| + | |style="text-align:right;"|ואמנם אשר תחת ההויה מהם הנה התמורה תקרה להם בעצם ובטבע | ||
| + | |- | ||
| + | | | ||
| + | :The sphere and what it contains are affected by the movement in the place. | ||
| + | |style="text-align:right;"|ואמנם הגלגל ומה שבו הנה תקרה להם ההעתקה במקום | ||
| + | |- | ||
| + | | | ||
| + | :The fire, the air, the water and the earth are affected by the shifting and the change in part, not in totality. | ||
| + | |style="text-align:right;"|ואמנם האש והאויר והמים והארץ יקרה להם ההעתק והתמורה בקצת בלתי הכל | ||
| + | |- | ||
| + | |Each one of those, whose change was described, is affected by it during its whole existence. | ||
| + | |style="text-align:right;"|וכל אחד מאלו אשר תארנום במה שיקרה להם מהתמורה הנה הם בו כל ימי היותם | ||
| + | |- | ||
| + | |By his saying "the first property of the primeval matter", he meant the individuation of the individuals, who are transformed from a non-being into a being, and "the element" is the potentiality. | ||
| + | |style="text-align:right;"|ואמרו הסגלה הראשונה מן ההיולי ירצה בו איוש המתאיישים שהם יומרו מה שהיו מלא אל יש והיסוד הוא האפשרות | ||
| + | |- | ||
| + | |'''The author of this book''' [= Nicomachus] '''said''': Those that are non-bodies, which are what exists, i.e. the nine categories of accidents, due to their defined separation, do not deviate from their quality, and do not change their essence, meaning, the secondary accidents, for they are apprehended together. | ||
| + | |style="text-align:right;"|<big>אמר מניח הספר</big> ואמנם אשר אינם גשמים והם מה שימצא ר"ל מאמרות התשעה המקרים הנה הם בגדר הפרדתם בלתי סרים מתכונתם ולא משתנים מענינם ירצה המקרים השיניים כי הם מושכלים יחד | ||
| + | |- | ||
| + | |Yet, the change and transformation that occur in these individual perceptible accidents - such as the whiteness of such, the movement of such - they are due to their participation in the substance that carries them, i.e. when they subsist, they are affected by the change and transformation of it. | ||
| + | |style="text-align:right;"|אבל מה שישיג אישי אלו המקרים המוחשים כמו לובן פלני ותנועת פלני מהשנוי והתמורה הנה הם להשתתפם לעצם הנושא אותם ר"ל כשהם עומדים ישיגם השינוי והתמורה בתמורתו ושינויו | ||
| + | |- | ||
| + | |Because of this, the knowledge of them is not called science. | ||
| + | |style="text-align:right;"|ולזה ידיעתם לא תקרא חכמה | ||
| + | |- | ||
| + | |On the other hand, the secondary substances and the secondary accidents are those whose particular knowledge is the science. | ||
| + | |style="text-align:right;"|אבל העצמים השניים והמקרים השניים הנה הם אשר ידיעתם בפרט היא החכמה | ||
| + | |- | ||
| + | |Although, the knowledge of the individuals is called science metaphorically, since it is the path towards the knowledge of those permanent, eternal and primary things, which are not subject to change nor to removal of their nature, and which are said to exist truly and distinctly. | ||
| + | |style="text-align:right;"|ואמנם על ההעברה הנה תקרא ידיעת האישים חכמה אחר שהיא הדרך אל ידיעת אותם הדברים המתמדיים הנצחיים הראשונים אשר לא ישיגם שנוי ולא הסרה מטבעם אשר יאמר שהם נימצאים באמת ובברור | ||
| + | |- | ||
| + | |But, the individuals that are subject to the six movements, which are the generation and corruption, the growth and decline, and the change and displacement, they are said to exist because of the existence of their species, since, as explained, they give them their names and definitions. | ||
| + | |style="text-align:right;"|אבל האישים הנופלים תחת התנועות השש אשר הן ההויה וההפסד והגדול והחסרון וההשתנות וההעתק הנה אמנם יאמרו שהם נימצאים מפני מציאות מיניהם אחר שמיניהם כמו שבארנו יתנו להם שמותיהם וגדריהם | ||
| + | |- | ||
| + | |Indeed, when we aim at one of their individuals, there is no being having its nature, since it does not exist, even for a single moment, in one and the same property, for the individuals, at any time, are in motion and in transformation. | ||
| + | |style="text-align:right;"|ואמנם כאשר נכוון אל אחד מאישיהם לא ימצא בטבעו נמצא לפי שלא יתקיים כהרף עין אל עניין אחד אחר שהאישים בכל עת להם תנועה ותמורה | ||
| + | |- | ||
| + | |Because of this, ''<span style=color:blue>Plato</span>'' said in ''<span style=color:blue>Timaeus</span>'': What is the thing that always exists, without ever becoming? And what is the thing that always becomes without ever being? | ||
| + | |style="text-align:right;"|ולזה אמר {{#annot:Plato|509|OIzu}}אפלטון{{#annotend:OIzu}} ב{{#annot:Timaeus|2650|sgP8}}ספר טימאוש{{#annotend:sgP8}} מה הדבר אשר הוא נמצא לעולם ואין לו התהוות כלל ומה הדבר אשר יתהווה לעולם ואין לו מציאות כלל | ||
| + | |- | ||
| + | |By the first, he meant the species and genera that are homogeneous eternally, which are not subject to generation, and which are indeed apprehended by the intellect, i.e. by guidance from what is perceptible. | ||
| + | |style="text-align:right;"|ירצה בראשון הסוגים והמינים המתדמים לנצח אשר לא תשיגם הויה אמנם יושגו בשכל ר"ל בהדרכה מהמוחשות | ||
| + | |- | ||
| + | |By the second, he meant the individuals that are thought to exist at one time, but which are not really so, because they are subject to generation and transformation, without being of the same property at two different times. | ||
| + | |style="text-align:right;"|ואמנם השני ירצה בו האישים אשר יחשב שהם נימצאים בעת אחד ואינם כן באמת אחר שיתהוו ויומרו מבלתי שימצאו בשני עיתים בענין אחד | ||
| + | |- | ||
| + | |'''He''' [= Nicomachus] '''said''': It is appropriate for us and necessary to us, if we desire the perfection of man - as far as it is possible - and the permanent survival, that it will be achieved by philosophy alone not by anything else. | ||
| + | |style="text-align:right;"|<big>אמר</big> הנה כבר ראוי לנו ויחוייב עלינו אם היינו נכספים לשלמות האדם כפי יכלתו וההשארות המתמיד והיה זה אמנם יהיה בפילוסופיה לבדה לא בדבר זולתה | ||
| + | |- | ||
| + | |Philosophy is, as we have mentioned, the love of science. | ||
| + | |style="text-align:right;"|והפילוסופיה כמו שזכרנו אהבת החכמה | ||
| + | |- | ||
| + | |Science is the veracious knowledge of existing things. | ||
| + | |style="text-align:right;"|והחכמה אמיתות הידיעה בדברים הנימצאים | ||
| + | |- | ||
| + | |Among the existing things, some are said to truly exist, and some are called such by homonymy. | ||
| + | |style="text-align:right;"|והדברים הנימצאים מהם מה שיאמר לו נמצא באמת ומהם מה שיאמר זה בשתוף השם | ||
| + | |- | ||
| + | |It is necessary to examine the existing things and explain them according to the truth. | ||
| + | |style="text-align:right;"|מחוייב שנחקור מהדברים הנמצאים ונבארם באמתות | ||
| + | |- | ||
| + | |We say that among the perceptible existences, there are those whose parts are unified, their members are integrated - this is as the rank of the animal, whose parts are unified in it, or the tree, and their like - those are called magnitudes truthfully. | ||
| + | |style="text-align:right;"|ונאמר שהנמצאות המוחשות מה שהם מתאחדי החלקים מחוברי האיברים וזה כמדרגת החי אשר חלקיו מתאחדים בו וכמו כן האילן והדומה להם ואילו יאמר להם גדלים באמתות | ||
| + | |- | ||
| + | |And among them are those that are dissociated, so that when they are close in places and when they gather, they are called sums and multitudes - even though their parts are not unified - like the herds of goats and the rows of people. | ||
| + | |style="text-align:right;"|ומהם מה שהם מפורדים והם בהתקרב מקומותיהם ובהתקבצם יקראו כללים ורבויים ואם לא יהיו מתאחדי החלקים כמו עדרי הצאן ושורות האנשים | ||
| + | |- | ||
| + | |It has thus been clarified that, of the perceptible things, some are subject to magnitudes and some of them are subject to plurality. | ||
| + | |style="text-align:right;"|הנה כבר התבאר שמהמוחשים מהם מה שהוא נופל תחת הגודל ומהם מה שהוא נופל תחת הרבוי | ||
| + | |- | ||
| + | |Yet, these two species are infinite by nature, generally speaking. | ||
| + | |||
| + | :*Magnitude – begins with a finite whole and is divided endlessly | ||
| + | |style="text-align:right;"|אלא ששני אלו המינים אין תכלית להם בטבע כאשר יאמרו מאמר משולח | ||
| + | |- | ||
| + | |For, the plurality, starting from a limited origin and multiplied, does not have by nature an end at which it would stop, and it does not multiply a single time, but it always continues to grow. | ||
| + | |style="text-align:right;"|כי הרבוי כאשר יתחיל משרש מוגבל ויכפיל לא יהיה לו תכלית בטבע יעמד אצלו ולא יהיה לו כפל אחד אבל יוסיף ויצמח תמיד | ||
| + | |- | ||
| + | |Likewise, the magnitude, beginning its division from a limited totality, the division does not stop at an indivisible part, but always admitting division. | ||
| + | |style="text-align:right;"|וכמו כן הגודל כאשר יתחיל בחלוקתו מכללות מוגבל לא תכלה החלוקה אל חלק אין חלק לו אבל יסבול החלוקה תמיד | ||
| + | |- | ||
| + | |Therefore, the knowledge of these two does not really exist, because the knowledge of the infinite does not exist. | ||
| + | |style="text-align:right;"|הנה אין ידיעת שני אלו נמצאת על האמת אחר שידיעת מה שאין תכלית לו בלתי נמצאת | ||
| + | |- | ||
| + | |Indeed, true knowledge is the knowledge of circumscribed limited things. | ||
| + | |style="text-align:right;"|ואמנם הידיעה על אמיתתו ידיעת הדברים הנכללים המוגבלים | ||
| + | |- | ||
| + | |It has thus been clarified that the knowledge does not recognize the absolute plurality, because it is infinite, and what is infinite is unlimited, and what is unlimited there is no way whatsoever to its knowledge. | ||
| + | |style="text-align:right;"|הנה כבר התבאר שהידיעה לא תעמד אצל הרבוי המשולח לפי שהוא בלתי בעל תכלית ומה שהוא בלתי בעל תכלית הוא בלתי מוגבל ומה שהוא בלתי מוגבל אין דרך אל הידיעה בו כלל | ||
| + | |- | ||
| + | |However, if we speak of the plurality in relation to the less and we call the two related terms by their specific names, then they are knowable: | ||
| + | |style="text-align:right;"|ואמנם אם נאמר הרבוי בהצטרף אל המיעוט ונקרא שני המצטרפים בשמותם המיוחדים היו אז ידועים | ||
| + | |- | ||
| + | | | ||
| + | :The many is many compared to what is less than it, and the few is few compared to what is more than it. | ||
| + | |style="text-align:right;"|לפי שהרב אמנם יהיה רב אצל מה שהוא פחות ממנו וכמו כן המעט אמנם הוא מעט אצל מה שהוא יותר ממנו | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :The few, compared to what is more than it, is more compared to what is less than it. |
| + | |style="text-align:right;"|והמעט אצל מה שהוא יותר ממנו הוא הרב אצל מה שהוא פחות ממנו | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::For example, the ten is more compared to what less than it, and few compared to what is more than it. |
| + | |style="text-align:right;"|כמו העשרה שהוא הרבה כשהוקש אל מה שתחתיו ומעט כשהוקש אל מה שעליו | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Likewise, the great is great, when it is compared to what is smaller than it, but, when it is compared to what is greater than it, it is small. |
| + | |style="text-align:right;"|וכן הגדול אמנם הוא גדול כשהוקש אל מה שהוא יותר קטן ממנו ואם הוקש אל מה שהוא יותר גדול ממנו היה קטן | ||
| + | |- | ||
| + | |It has thus been clarified from these two aspects that, although they are unlimited by themselves, they are nevertheless limited when they are in relation. | ||
| + | |style="text-align:right;"|הנה כבר התבאר משני אלו הצדדים שאפלו היו בלתי מוגבלים בעצמם הנה הם עם ההצטרף אליהם מוגבלים | ||
| + | |- | ||
| + | |Let us now say that the numerical quantity is divided into two categories: | ||
| + | |style="text-align:right;"|ונאמר עתה ש{{#annot:term|2580,2098|6gVR}}הכמה המספרי{{#annotend:6gVR}} יחלק לשני חלקים | ||
| + | |- | ||
| + | | | ||
| + | :1) One is that, of which what is ascribed to it, is ascribed to it in itself and by nature. | ||
| + | |style="text-align:right;"|אחד מהם הוא אשר מה שחוייב לו יחוייב לו לבדו ובטבעו | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :2) The other is that, of which what is ascribed to it, is ascribed to it relatively. |
| + | |style="text-align:right;"|והאחר הוא שמה שיחוייב לו יחוייב לו בהצטרף | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|אמר מניח הספר ויורה על מלאכת החשבון ג"כ יותר קודמת בטבע מזולתה שהיא תשים זולתה מאלו החכמות אובד באבדה מבלתי שתאבד היא באבדן דבר מהן כי אם יסתלק המספר | + | *The ascribed to it by nature, such as: the even and the odd, the even-times-even, the even-times-odd; the first incomposite odd, and the second composite. |
| + | |style="text-align:right;"|אמנם המחוייב לו בטבע כמו הזוג והנפרד וזוג הזוג וזוג הנפרד והנפרד הראשון בלתי המורכב והשני המורכב | ||
| + | |- | ||
| + | | | ||
| + | :As these are ascribed to the numerical quantity by its nature, not by its relation to another. | ||
| + | |style="text-align:right;"|כי אלו אמנם יחוייבו לכמה המספרי בטבעו לא מפני הצטרפו אל זולתו | ||
| + | |- | ||
| + | | | ||
| + | ::For, the even is not divisible into two parts of equal units due to its relation to another thing. | ||
| + | |style="text-align:right;"|כי אין הזוג מתחלק לשני חלקים מתמשלי האחדים מפני הצטרפו אל דבר אחר | ||
| + | |- | ||
| + | | | ||
| + | *The ascribed to it, that is ascribed to it by relation, such as: the double, the half and the third. | ||
| + | |style="text-align:right;"|ואמנם אשר יחוייב לו מה שיחויב בהצטרף כמו הכפל והחצי והשליש | ||
| + | |- | ||
| + | | | ||
| + | ::For the half is a half of something, and the double is double of something. | ||
| + | |style="text-align:right;"|כי החצי לדבר מה חצי והכפל לדבר מה כפל | ||
| + | |- | ||
| + | |It has, therefore, been clarified that the quantity is a science investigated by two arts: | ||
| + | |style="text-align:right;"|הנה אם כן התבאר שהכמה חכמה יחקרוה שתי מלאכות הם | ||
| + | |- | ||
| + | | | ||
| + | :'''<span style=color:green>[1. Arithmetic – investigation of the absolute quantity]</span>''' | ||
| + | *Arithmetic, which investigates the absolute quantity. | ||
| + | |style="text-align:right;"|הארתמאיתיקא והיא תחקור מ{{#annot:term|34,1336|jC7h}}הכמה הניפרד{{#annotend:jC7h}} | ||
| + | |- | ||
| + | | | ||
| + | :'''<span style=color:green>[2. Music – investigation of the relative quantity]</span>''' | ||
| + | *Music, which investigates the relative quantity. | ||
| + | |style="text-align:right;"|והמוסיקא והיא תחקור מהכמה המצורף | ||
| + | |- | ||
| + | |'''<span style=color:green>[The science of quality is investigated by two arts:]</span>''' | ||
| + | The quality of natural things is divided into two categories: quality of the moving and quality the resting. | ||
| + | |style="text-align:right;"|ואמנם איכות הדברים הטבעיים יחלק לשני חלקים אם איכות מתנועע ואם איכות נח | ||
| + | |- | ||
| + | | | ||
| + | :'''<span style=color:green>[1. Astronomy – investigation of what is moving]</span>''' | ||
| + | *The moving is what is investigated in the art of astronomy. | ||
| + | |style="text-align:right;"|והמתנועע הוא מה שיחקור במלאכת האסטרונומייא ר"ל התכונה | ||
| + | |- | ||
| + | | | ||
| + | :'''<span style=color:green>[2. Geometry – investigation of what is resting]</span>''' | ||
| + | *The resting is what is investigated in the art of geometry. | ||
| + | |style="text-align:right;"|והנח הוא מה שיחקר במלאכת הגימטריא ר"ל ההנדסה | ||
| + | |- | ||
| + | |'''He''' [= Nicomachus] '''said''': There is no other way to arrive at the knowledge of the species that have been said to truly exist, except through these four arts which are: arithmetic, geometry, astronomy and music. | ||
| + | |style="text-align:right;"|<big>אמר</big> הנה אין דרך אל ידיעת מיני מה שנאמר שהם נימצאים באמת רק באלו האומניות הד' אשר הם הארתמאיטיקא וההנדסה והתכונה וחבור הנגונים | ||
| + | |- | ||
| + | |There is no path leading to philosophy except through them. | ||
| + | |style="text-align:right;"|ולא ימצא דרך אל הפילוסופיה אלא בם | ||
| + | |- | ||
| + | |As, in every art, the practitioner must have the expertise in his art and needs a model which directs him in the execution of his plan, so are these sciences for the art of the philosophy, which is the art of the arts. | ||
| + | |style="text-align:right;"|וכמו שכל מלאכה מן המלאכות יצטרך עושה אל בקיאות במלאכתו ודמיון יתישר ממנו בהוצאת דרושו כן אלו החכמות במלאכת הפילוסופיה אשר היא מלאכת המלאכות | ||
| + | |- | ||
| + | |This has already been said by ''<span style=color:blue>Androcydes the Pythagorean</span>''. | ||
| + | |style="text-align:right;"|וכבר יאמר זה המאמר אנדרוקודיס הפיתאגוריי | ||
| + | |- | ||
| + | |''<span style=color:blue>Archytas of Taranto</span>'', too, at the beginning of his Art of the Composition of Melodies, also said: The ancients did well to see from these sciences, that it is by no means impossible that they will have for everything a unique and true opinion. | ||
| + | |style="text-align:right;"|וכן יאמר גם כן ארגוטאלס אל אטראנטיני כאשר התחיל במלאכת חבור הליחנים שהוא אמר הטיבו הראשונים במה שראו מענין אלו החכמות כי אינו בלתי אפשר כלל שיהיה להם בכל דבר סברה אחת אמיתית | ||
| + | |- | ||
| + | |Just as they excel in the nature of the whole, they were willing to have a true opinion on each of the parts. | ||
| + | |style="text-align:right;"|הנה כמו שהם כבר הטיבו במה שראו מענין טבע הכל כן היו מוכנים לסבור בכל אחד מן החלקים סברא אמיתית | ||
| + | |- | ||
| + | |For they offered us in the sections of geometry and astronomy a clear and obvious science, and they were no less useful in giving us the virtues of the art of music. | ||
| + | |style="text-align:right;"|כי הם הקנו לנו בשערי המדות והתכונה חכמה גלויה ומבוארת ולא קצרו גם כן מזה במה שהועילונו מעלות מלאכת הלחנים | ||
| + | |- | ||
| + | |These sciences can be imagined as four brothers. | ||
| + | |style="text-align:right;"|וכבר ידומה שאלו החכמות הארבעה אחים | ||
| + | |- | ||
| + | |Yet, they are reducible to the first two species, namely the quantity and the quality, as explained previously. | ||
| + | |style="text-align:right;"|ואם היו כבר ישובו אל שני המינים הראשונים אשר הם הכמות והאיכות כמו שביארנו לפנים | ||
| + | |- | ||
| + | |Similarly, ''<span style=color:blue>Plato</span>'', at the end of the thirteenth treatise of the book he wrote on laws, which is the book that people call the "Philosophy", examining and defining what is proper for a true philosopher to be in the knowledge of these four, summarized it, after having explained it at long, saying that any shape, sum of numbers, harmonic music, and what is indicated by the movements of the planets, its knowledge should necessary be practice individually by the measure of their harmony. | ||
| + | |style="text-align:right;"|עם ש''אפלטון'' בסוף האופן השלשה עשר מהספר אשר הניח בנימוסים והוא הספר אשר יקראוהו האנשים הפילוסופיא בחקרו וגדרו איך ראוי שיהיה אשר הוא פילוסוף באמת כן בידיעת אלו הארבעה אחר כן שב ואמר בקצרה אחר שהפליג בזה והקדים שכל תמונה וקיבוץ מספר והסכמת חבור ומה שיורו בו ממרוצות הכוכבים אמנם ראוי שיורגל ידיעתו כפי שעור חבורם אחד אחד | ||
| + | |- | ||
| + | |The cause of what is mentioned is clear: when man intensively learns to apprehend through them one known, which he expects of them, they aim at one thing, even though they are several. | ||
| + | |style="text-align:right;"|וכבר יתבאר עלת מה שזכרנוהו כי כאשר היה האדם אמנם יתלמד הרבה להשיג בם ידוע אחד הוא כונתו מהם הנה אמנם הם דבר אחד ואם רבו | ||
| + | |- | ||
| + | |However, if man exchange this path by the science of philosophy, he will not reach it, since it is the way in which the path leading to the existence is reached, there is no other way at all, i.e. the four mentioned sciences, whether difficult or easy, numerous or few. | ||
| + | |style="text-align:right;"|ואמנם אם יחליף האדם זה הדרך בחכמת הפילוסופיא אין דרך לו אליה לפי שזה האופן אשר ממנו יבא הדרך אשר ממנו המציאות אין דרך לו זולת כלל ר"ל החכמות הארבעה אשר זכרנו קשות היו או קלות רבות היו או מועטות | ||
| + | |- | ||
| + | |Their acquisition should not be neglectful, for who has united these sciences in the described mode, is the one whom I call a true scholar and describe as indulging in his science. | ||
| + | |style="text-align:right;"|אין ראוי להתרשל בקניניהם כי מי שקיבץ אלו החכמות על צד שתארתי הוא אשר אקראהו אני חכם אמתי ואתארהו שש בחכמתו | ||
| + | |- | ||
| + | |It is not hidden that these sciences are like bridges through which our thoughts pass from those perceptible apprehended things to the existing intelligible things, they transfer our thoughts from those corporeal things, with which we have grown and which are familiar to us, to things which are foreign to us, which are not familiar to our senses, and which, by their subtlety, are similar to our souls. | ||
| + | |style="text-align:right;"|כי לא יעלם שאלו החכמות הם כמו גשרים בם יעברו מחשבותינו מאלו הדברים המוחשים המחושבים אל הדברים הנימצאים המושכלים ויעבירו דעותנו מאלו הדברים הגשמיים אשר גדלנו בם והרגלנום אל הדברים הזרים אצלנו אשר לא הרגילום חושינו ואשר הם בדקותם דומים לנפשותינו | ||
| + | |- | ||
| + | |The best suited of what was chosen in the introduction to the investigation of the sciences and their acquisition is what ''<span style=color:blue>Plato</span>'' mentioned in '''the Book of Laws''' that Socrates told him, and he tries to be clever by putting forward true and useful reasons for these sciences that are necessary for the sciences that are useful for the improvement of human lives, for he said: | ||
| + | |style="text-align:right;"|והיותר ראוי ממה שבחרנוהו בהקדמת העיון בחכמות וקנינם מה שזכרו ''אפלאטון'' בספר הנימוסים שסקראט אמר לו והוא יתחכם כשיביא עלות אמיתיות מועילות בענין אלו החכמות מתחייבות מצד החוכמות מועילות בתיקון חיי בני אדם אחר שיאמר | ||
| + | |- | ||
| + | | | ||
| + | *Arithmetic is needed for calculations, sharing, harvesting, spending, repayment of loans, and for partnerships. | ||
| + | |style="text-align:right;"|אמנם מלאכת החשבון אמנם נצטרך אליה בחשבונות או בחלוקות ובתבואות ובהוצאות ופרעון החובות והשותפיות | ||
| + | |- | ||
| + | | | ||
| + | *Geometry - for erecting encampments, building cities and palaces, and measuring fields. | ||
| + | |style="text-align:right;"|אמנם המדות בהשואת המחנות ובניין המדינות וההיכלות ומדידת השדות | ||
| + | |- | ||
| + | | | ||
| + | *Astronomy - knowing the times for the cultivation of lands, for the navigation at sea, and for other choices concerning the times favorable to the implementation of certain arts and the seasons. | ||
| + | |style="text-align:right;"|אמנם חכמת הכוכבים בידיעת עיתות עבודת הקרקעות ורכיבת הים וזולת זה מבחירות עיתות התחלות המלאכות ופרק הזמנים | ||
| + | |- | ||
| + | | | ||
| + | *Music - thanking the exalted Creator, or rejoicing in choruses or privately. | ||
| + | |style="text-align:right;"|אמנם חכמת הניגונים להודות בם הבורא ית' או לשמוח במקהלות או בצניעה | ||
| + | |- | ||
| + | |To this ''<span style=color:blue>Plato</span>'' replied, rebuking him: do you think, seeing that I am indulgent towards you, that I will not dispute you? | ||
| + | |style="text-align:right;"|ולזה יאמר אפלאטון משיב לו והוא יוכיחהו התחשבני כי תראה שאחמול עליך שלא אחלק עמך | ||
| + | |- | ||
| + | |I say that these sciences can not be doubted; for this dispute would be quite difficult from every side. | ||
| + | |style="text-align:right;"|הנני אומר שאלו החכמות אין לדקדק בם שזה החלוק יקשה מאד מכל צד | ||
| + | |- | ||
| + | |But, I will add support: there is no way to say that the eye of the soul, i.e. when you are blinded and other corporeal activities are closed off, spreading a veil over you, it is opened and awakened in these sciences. | ||
| + | |style="text-align:right;"|אבל אוסיפך חזוק שאין דרך לאחד לומר זה כי עין הנפש ר"ל כאשר יעצומוהו ויסתמוהו שאר הפעולות הגופיות וישימו עליך מסך הנה הוא יפקח ויעור באלו החכמות | ||
| + | |- | ||
| + | |The truth of the eye of the soul is more excellent than the truth of ten thousand bodily eyes, for it is only through it that the truth of things can be seen. | ||
| + | |style="text-align:right;"|ואמתת עין הנפש יותר משובח מאמתת עשרת אלפים עינים מהעינים הגופים כי בו לבדו יראו אמתויות הדברים | ||
| + | |- | ||
| + | |<span style=color:red>The order of the natural sciences</span><br> | ||
| + | '''He''' [= Nicomachus] '''said''': The first of these four sciences, the one which is anterior to the others by nature, the one that is best established, that is for them in the rank of the generative, from which the beginning of their existence and the root of their growth, is the art of arithmetic. | ||
| + | |style="text-align:right;"|<big>אמר</big> והיותר ראשונה מאלו החכמות הארבעה והיותר קודמת על הנשארות בטבע והיותר חזקת האמונה והיא להם כמדרגת המולידה אשר ממנה התחלה היותן ושרש צמיחתן הוא מלאכת החשבון | ||
| + | |- | ||
| + | |It is not because that it is that which will exist in the government of the Creator, but because it was, in a rank of an allegory, first in the thought of God, and the metaphor, from which are derived the properties of the things which the exalted produced from the foundation and which he has completed according to the specific properties of each. | ||
| + | |style="text-align:right;"|אין זה לפי שהיא היא אשר תתקיים בהנהגת הבורא אבל לפי שהיא במדרגת ההמשל היתה ראשונה במחשבת האל והדמיון אשר ממנו יקחו תכונת הדברים אשר הצמיחם ית' מהיסוד והשלימם על הענינים המיוחדים אשר עליהם כל אחד מהם | ||
| + | |- | ||
| + | |'''The author of this book''' [= Nicomachus] '''said''': what indicates that the art of arithmetic is, by nature, anterior to others, is that its disappearance would lead to the disappearance of others, while the disappearance of one of these would not lead to its disappearance. | ||
| + | |style="text-align:right;"|<big>אמר מניח הספר</big> ויורה על מלאכת החשבון ג"כ יותר קודמת בטבע מזולתה שהיא תשים זולתה מאלו החכמות אובד באבדה מבלתי שתאבד היא באבדן דבר מהן | ||
| + | |- | ||
| + | |For, if the number disappears, the counted disappear; but, if they the counted disappear, the number does not disappear. | ||
| + | |style="text-align:right;"|כי אם יסתלק המספר יעדרו הספורים ולא אם יעדרו הספורים יאבד המספר | ||
| + | |- | ||
| + | | | ||
| + | ::As we say about the animal that it is anterior to the man by natural precedence, because when the animal disappears, the man disappears with its disappearance, but not that when the man disappears, the animal disappears. | ||
| + | |style="text-align:right;"|כאמרנו בחי שהוא יותר קודם מן האדם קדימה טבעית שכאשר יעלה החי יעלה האדם בהעלותו ולא כשיעלה האדם יעלה החי | ||
| + | |- | ||
| + | | | ||
| + | :So for any thing that is anterior by natural precedence to another thing, when the anterior disappears, the other disappears with its disappearance, but it does not disappear with the disappearance of what is posterior to it. | ||
| + | |style="text-align:right;"|וכן כל דבר יותר קודם מדבר אחר קדימה טבעית כשיעלה הקודם יעלה בהעלותו זולתו ולא יעלה הוא בהעלות דבר ממה שהוא יותר קודם ממנו | ||
| + | |- | ||
| + | | | ||
| + | :Also when reversing the statement, the same meaning is found: when a thing is necessary, its necessity implies the necessity of what is anterior to it by natural precedence; but, when the anterior is necessary, its necessity does not imply the necessity of what is below it in precedence. | ||
| + | |style="text-align:right;"|וכן כאשר תהפך המאמר תמצא הענין כפי זה כשיחויב דבר יחויב בחיובו מה שהוא יותר קודם ממנו קדימה טבעית ולא כשיחויב הקודם יהיה הכרח שיחויב בחיובו מה שהוא תחתיו בקדימה | ||
| + | |- | ||
| + | | | ||
| + | ::As the man and the poet, for the man is anterior to the poet, and when the poet is necessary, the man is necessary; but if the man is necessary it is not necessary that the poet is forcibly. | ||
| + | |style="text-align:right;"|כמו האדם והמשורר כי האדם יותר קודם מהמשורר וכשיחויב משורר יחויב שיהיה אדם ואין הכרח כשיחויב אדם שיהיה בלי ספק משורר | ||
| + | |- | ||
| + | | | ||
| + | *Thus, according to this order of these four aforementioned sciences, when geometry exists, no doubt arithmetic exists with it. | ||
| + | |style="text-align:right;"|הנה כפי זה הסדר אלו החכמות הארבעה אשר זכרנו כאשר תהיה המדידה אין ספק שיהיה החשבון עמה | ||
| + | |- | ||
| + | | | ||
| + | ::Indeed, when, a triangle, a quadrilateral, an octahedron, or a pentadecagon are necessary in geometry, the art of arithmetic is already used, since the four, the three, the eight and the fifteen come from arithmetic. | ||
| + | |style="text-align:right;"|כי כשיחויב במדות משולש ומרובע ובעל שמנה תושבות או חמש עשרה תושבות הנה כבר השתמשת בזה ממלאכת החשבון לפי שהארבעה והשלשה והשמנה והט"ו מן החשבון | ||
| + | |- | ||
| + | | | ||
| + | :Arithmetic is not absent from geometry, nor are numbers that are necessarily required when it is required. | ||
| + | |style="text-align:right;"|ולא תמצא מלאכת המדות ריקה מן החשבון והמספרים אשר יחויבו בחיובה בהכרח | ||
| + | |- | ||
| + | | | ||
| + | ::For, there is no way to say multiplied three times and four times without the knowledge of the three and the four before by assumption. | ||
| + | |style="text-align:right;"|כי אין דרך לומר בכפל שלשה פעמים וארבעה פעמים מבלתי ידיעת השלשה והארבעה קודם בהנחה | ||
| + | |- | ||
| + | | | ||
| + | ::On the other hand, reversing the statement, one finds that the three, the four, and the other numbers already exist in nature and in thought, even if the magnitudes, whose names are derived from them, are not known or do not exist. | ||
| + | |style="text-align:right;"|ואמנם אם תהפך המאמר תמצא השלשה והארבעה וזולתה מהמספרים כבר נתקיימו בטבע והמחשבה ואם לא היו הגדלים הנגזרי השמות מהם ידועים או נמצאים | ||
| + | |- | ||
| + | | | ||
| + | :It has thus been proved that the number is anterior to the measures by a natural precedence. | ||
| + | |style="text-align:right;"|הנה כבר התבאר אם כן שהמספר יותר קודם מן המדות קדימה טבעית | ||
| + | |- | ||
| + | | | ||
| + | :Since when the arithmetic disappear, the geometry also disappears, but the arithmetic does not disappear with its disappearance. | ||
| + | |style="text-align:right;"|אחר שבסור החשבון תסור המדידה ולא יסור החשבון בסורה | ||
| + | |- | ||
| + | | | ||
| + | *Likewise, by a similar explanation it is clear that the art of arithmetic is anterior to music. | ||
| + | |style="text-align:right;"|וכן גם כן בכמו זה הבאור יתבאר שמלאכת החשבון יותר קודמת ממלאכת הלחנים | ||
| + | |- | ||
| + | | | ||
| + | :Since the substance is anterior to the relation: | ||
| + | |style="text-align:right;"|לפי שהעצם יותר קודם מההצטרפות | ||
| + | |- | ||
| + | | | ||
| + | :*As the money is anterior to the abundance of the money and the substance is anterior to the magnitude of the substance. | ||
| + | |style="text-align:right;"|כמו שהממון יותר קודם מרבוי הממון והעצם יותר קודם מגודל העצם | ||
| + | |- | ||
| + | | | ||
| + | :*And the man is anterior to the instruments. | ||
| + | |style="text-align:right;"|והאדם יותר קודם מהכלים | ||
| + | |- | ||
| + | | | ||
| + | :This is because the composition of the melodies, their affinity and their harmony, are when the term of one sound of them is in accordance with the other. | ||
| + | |style="text-align:right;"|וזה לפי שחבור הנגונים והאותותם והסכימם אמנם הוא כשיהיה תכלית הקול אחד מהם אצל האחר | ||
| + | |- | ||
| + | | | ||
| + | ::This by the harmony of the quarter, of the fifth, of each three times [= triple ratio], and of each four times [= quadruple ratio]. | ||
| + | |style="text-align:right;"|וזה בהסכמה אשר בארבעה ואשר בחומש ואשר בכל שלשה פעמים ואשר בכל הארבעה פעמים | ||
| + | |- | ||
| + | | | ||
| + | ::By each combination of ratios [= proportion] we mean the number of sounds it contains. | ||
| + | |style="text-align:right;"|וכל קבוץ יחסים אמנם נרצה ממנו מספר הנעימות אשר בו | ||
| + | |- | ||
| + | | | ||
| + | ::*What is in the four [quarta]: one of its terms is to the other as its whole and its third. [diatessaron <math>\scriptstyle{\color{blue}{4:3=1+\frac{1}{3}}}</math>] | ||
| + | |style="text-align:right;"|ואשר בארבעה אחד מתכליותיו אצל האחר כמוהו וכמו שלישיתו | ||
| + | |- | ||
| + | | | ||
| + | ::*What is in the five [quinta]: one of its terms is to the other as its whole and its half. [diapente <math>\scriptstyle{\color{blue}{3:2=\frac{1}{2}}}</math>] | ||
| + | |style="text-align:right;"|ואשר בחמשה אחד מתכליותיו אצל האחד כמהו וכמו חציו | ||
| + | |- | ||
| + | | | ||
| + | ::*What is in the four and in the five: the ratio of both terms is that of the whole, one term being to the other in the double ratio; and one of the terms is to the other in the triple ratio. | ||
| + | |style="text-align:right;"|ומאשר בארבעה ואשר בחמש יהיו שני התכליות ביחס אשר בכל ואשר אחד מתכליותיו אצל האחר כפלו ואשר בכל ואשר אחד מתכליותיו אצל האחר שלשה דמיוניו | ||
| + | |- | ||
| + | | | ||
| + | ::*for the term of the whole and five is to the other as the ratio of the whole and half of the whole. [diapente <math>\scriptstyle{\color{blue}{3:2=1+\frac{1}{2}}}</math>] | ||
| + | |style="text-align:right;"|לפי שתכלית אשר בכל וחמש אצל אשר בכל ביחס כל וחצי כל | ||
| + | |- | ||
| + | | | ||
| + | ::*and in four and in each one of its terms is to the other as [four] is to three. [diatessaron <math>\scriptstyle{\color{blue}{4:3}}</math>] | ||
| + | |style="text-align:right;"|ואשר בארבעה ובכל אחד מתכליותיו אצל האחר כשמנה אצל השלשה | ||
| + | |- | ||
| + | | | ||
| + | ::*In every four times is such that one of its terms is to the other its four times. | ||
| + | |style="text-align:right;"|ואשר בכל ארבעה פעמים הוא אשר אחד מתכליותיו אצל האחר ארבעה דמיוניו | ||
| + | |- | ||
| + | | | ||
| + | :These are all the combinations of music - they could not be combined without the existence of arithmetic. | ||
| + | |style="text-align:right;"|ואלו כל קבוצי החבור אין דרך לחברם והחשבון בלתי נמצא | ||
| + | |- | ||
| + | | | ||
| + | :Yet, when arithmetic exists, the combinations and melodies do not necessarily exist. | ||
| + | |style="text-align:right;"|וכשימצא החשבון לא יחוייב שיהיו החבורים ולא הלחנים נמצאים | ||
| + | |- | ||
| + | | | ||
| + | *By this explanation, it is also clear that the art of arithmetic is anterior to the art of astronomy. | ||
| + | |style="text-align:right;"|וכמו כן גם כן מלאכת החשבון בזה הבאור יתבאר שהיא קודמת ממלאכת התכונה | ||
| + | |- | ||
| + | | | ||
| + | :*This is obvious because all its suppositions are obtained from arithmetic and geometry. | ||
| + | |style="text-align:right;"|וזה גלוי אחר שבחשבון והמדות יושגו כל דרושיה | ||
| + | |- | ||
| + | | | ||
| + | :*It is also clear that geometry is anterior to astronomy, since movement follows rest. | ||
| + | |style="text-align:right;"|וכבר יתבאר גם כן שהמדה יותר קודמת מהתכונה מצד שהתנועה אמנם תהיה אחר המנוחה | ||
| + | |- | ||
| + | | | ||
| + | :*Moreover, it is said that the motions of the planets are harmonious melodies, i.e. they are in a simple harmonic relation, i.e. they are in the simplicity of the multiple ratio and the superparticular ratio; and since it is so, the musical harmony is anterior to astronomy. | ||
| + | |style="text-align:right;"|ועוד שכבר יאמר שתנועות הכוכבים הם נגונים נערכים ר"ל הן בייחס חבוריי פשוט ר"ל בפשיטות יחס בעל הכפלים ויחס המוסיף חלק וכאשר היה זה כן הנה החבור הנגוני יותר הקודם מהתכונה | ||
| + | |- | ||
| + | | | ||
| + | ::Indeed, it is not hidden that the risings of the planets, their declines, their eclipses, their occultation, and their visibility, are apprehended from the geometry. | ||
| + | |style="text-align:right;"|ואמנם זריחות הכוכבים ועריבותיהם וקדריותיהם והסתרתם והראותם לא יעלם שכבר יושב במדה | ||
| + | |- | ||
| + | |Since the art of arithmetic is anterior to the others, what is said before is applied to it, that the discussion about it and the statements concerning it are the first in order of priority, and that is what will be done from here. | ||
| + | |style="text-align:right;"|וכאשר היתה מלאכת החשבון יותר קודמת מזולתה בראוי אם כן ממנה מה שהקדמנו לפנים שהמאמר עליה ומשפטי הדבור בה יותר ראשונים בקדימה ואנחנו בזה מכאן | ||
| + | |- | ||
| + | |Here is my brother the completion of the introduction of this book, up to the number, as you had requested, so it is. | ||
| + | |style="text-align:right;"|הנה לך אחי שלמות פתיחת זה הספר כאשר שאלת עד מקום המספר ממנו והיה כן | ||
| + | |- | ||
| + | |May you succeed by all that the Creator has lavished upon you, and may He, by His grace, lead you to His will. Amen. | ||
| + | |style="text-align:right;"|ובכל מה שהטיב הבורא עליך מצליח והוא בחסדו יישירך לרצונו אמן | ||
|- | |- | ||
| | | | ||
| Line 42: | Line 581: | ||
|- | |- | ||
| | | | ||
| − | === | + | |
| + | === [Absolute Quantity - Arithmetic] === | ||
| | | | ||
|- | |- | ||
| | | | ||
| − | ==== The Nature of Number ==== | + | ==== The Discussion on the Nature of Number ==== |
| − | + | |style="text-align:right;"|<big>הדבור בטבע המספר</big> | |
| + | |- | ||
| + | |All things, whose composition is established by nature, are found to be subject to a numerical order | ||
| + | |style="text-align:right;"|כל הדברים שתיקן הטבע חבורם ימצאו נופלים תחת סדר המספר | ||
| + | |- | ||
| + | |Such as the elements, the times, the movements of the spheres, and the rest of the natural phenomena. | ||
| + | |style="text-align:right;"|כמו היסודות והזמנים ותנועות השמים ושאר התקונים הטבעיים | ||
| + | |- | ||
| + | |By this, the number is necessary at the rank of allegory and metaphor from which the thing is understood. | ||
| + | |style="text-align:right;"|הנה בזה ראוי שיהיה המספר במדרגת ההמשל והדמיון אשר ממנו יוקח הדבר | ||
| + | |- | ||
| + | |The exalted Creator has created it as first by nature. | ||
| + | |style="text-align:right;"|וחדשו הבורא ית' ראשון בטבע | ||
| + | |- | ||
| + | |Indeed, the composition of the number itself has no other order, as it is, in its quality, eternal by itself, not by any other. | ||
| + | |style="text-align:right;"|ואמנם חבור המספר עצמו אין סדר לו זולתו לפי שהוא בתכונתו נצחי בעצמו לא עומד בזולתו | ||
| + | |- | ||
| + | |But, since every compound is compounded out of different things undoubtedly and out of things that also exist, | ||
| + | |style="text-align:right;"|אבל לפי שכל מחובר אמנם הוא מחובר מדברים מתחלפים בלי ספק ומדברים הם גם כן נמצאים | ||
| + | |- | ||
| + | |(as, the non-existent - their composition is not imaginable, | ||
| + | |style="text-align:right;"|אחר שאשר אינם נמצאים אין לשער חבורם ולא אשר הם | ||
| + | |- | ||
| + | |and if they existed, but are not similar, it is impossible to compound them), | ||
| + | |style="text-align:right;"|ואפילו היו נמצאים אלא לפי שהם בלתי דומים אי אפשר חבורם | ||
| + | |- | ||
| + | |it follows that the compound things are compounded out of existing different suitable things | ||
| + | |style="text-align:right;"|הנה נמשך לזה שהדברים המחוברים אמנם יחוברו מדברים נמצאים מתחלפים נאותים | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *If they were not different, they would have been one, therefore would not need composition. |
| + | |style="text-align:right;"|לפי שאם לא יהיו מתחלפים הנה הם אחד לא יצטרכו אל חבור | ||
|- | |- | ||
| | | | ||
| − | ==== The Definition of Number and its | + | *If they were not suitable, they would not have been homogeneous, and if they would not have been homogeneous, they would not have been subject to composition. |
| + | |style="text-align:right;"|ואם לא יהיו נאותים לא יהיו מתדמים ואם לא יהיו מתדמים אלא שהם הפכיים לא יפול בם החבור | ||
| + | |- | ||
| + | |Thus, the number is also diverse and homogeneous like this, as it is compound. | ||
| + | |style="text-align:right;"|הנה המספר יהיה גם כן מתחלף ונאות כך אחר שהוא מחובר | ||
| + | |- | ||
| + | |This is true, since it has two primary diverse suitable homogeneous species, i.e. the even and the odd. | ||
| + | |style="text-align:right;"|וזה אמת אחר שבו שני מינים ראשונים מתחלפים נאותים דומים ר"ל הזוג והנפרד | ||
| + | |- | ||
| + | |For the exalted Creator has compounded them with their diversity in a complicated composition, which has no separation in it, as it will be clarified in what follows. | ||
| + | |style="text-align:right;"|כי הבורא ית' חברם עם חלופם חבור מסתבך אין הפרדה בו כמו שיתבאר זה במה שיבא אחר זה | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== The Discussion on the Definition of Number and its Categorization ==== | ||
| − | + | |style="text-align:right;"|<big>הדבור בגדר המספר וחלוקתו</big> | |
| + | |- | ||
| + | |The '''number''' as a figurative expression is a sum of units or a total quantity that consists of units. | ||
| + | |style="text-align:right;"|המספר בהעברה מן המאמר הוא קבוץ האחדים או כלל הכמות המורכב מהאחדים | ||
| + | |- | ||
| + | |The number is divided in a first categorization into two parts: one of them is the even number and the other is the odd number. | ||
| + | |style="text-align:right;"|והמספר יחלק חלוקה ראשונה אל שני חלקים אחד מהם הזוג והאחר הנפרד | ||
| + | |- | ||
| + | |{{#annot:definition|63|N8GS}} | ||
| + | *The '''even number''' is divisible into two equal parts with no mediator unit between them by which one exceeds the other. | ||
| + | |style="text-align:right;"|וה{{#annot:term|63|vAh2}}זוג{{#annotend:vAh2}} יחלק לשני חלקים שוים אין ביניהם אחדות אמצעי יעדיף בו אחד מהם על האחר{{#annotend:N8GS}} | ||
| + | |- | ||
| + | | | ||
| + | *The '''odd number''' is that which there is no way by which it will be divided into two equal parts, but when it is divided as close to equality as possible there is necessarily a unit by which one of its parts exceeds the other. | ||
| + | |style="text-align:right;"|והנפרד הוא אשר אין דרך אל שיחלק בשני חלקים שוים אבל בהכרח כשיחלק בשני חלקים בתכלית הקירוב מן השווי היה בין חלקיו אחדות יעדיף בו אחד משני חלקיו על האחר | ||
| + | |- | ||
| + | | | ||
| + | :this is the definition according to the ordinary conception. | ||
| + | |style="text-align:right;"|וזה הוא הגדר כפי מה שיראו ההמון | ||
| + | |- | ||
| + | |'''According to Pythagoras''': | ||
| + | |style="text-align:right;"|ואמנם כפי מאמר פיתאגוריש | ||
| + | |- | ||
| + | | | ||
| + | *The '''even number''' is that whose division demonstrates the extensive ratio of the beginning of decreasing and the beginning of increasing. | ||
| + | |style="text-align:right;"|הנה המספר הזוג הוא אשר יחלק חלוקה יראה בה היחס המקיף בתחלת המיעוט ותחלת הרבוי | ||
| + | |- | ||
| + | | | ||
| + | ::By the extensive ratio of the beginning of decreasing and the beginning of increasing he means the double ratio and subdouble ratio that are evident only in the division of the even number, i.e. the divisible into two equal parts. | ||
| + | |style="text-align:right;"|ירצה ביחס המקיף בתחלת המיעוט ותחלת הרבוי יחס הכפל והחצי אחר שלא יראה אלא בחלוקת המספר הזוג ר"ל המתחלק בשני חלקים שוים | ||
| + | |- | ||
| + | | | ||
| + | :'''He''' [= Nicomachus] '''said''': Indeed, the '''odd number''' is that which does not admit this, but is always divisible into two unequal parts. | ||
| + | |style="text-align:right;"|<big>אמר</big> ואמנם הנפרד הנה הוא אשר לא יקבל זה אבל הוא יחלק בשני חלקים בלתי שוים לעולם | ||
| + | |- | ||
| + | |'''According to ancients''' | ||
| + | |style="text-align:right;"|וכפי צד מאמר הראשונים גם כן | ||
| + | |- | ||
| + | | | ||
| + | *The '''even number''' is that which is divisible into two equal parts, and divisible also into two unequal parts by excess and deficiency. | ||
| + | |style="text-align:right;"|הנה המספר הזוג הוא אשר יחלק בשני חלקים שוים ויחלק גם כן בשני חלקים בלתי שוים בתוספת והחסרון | ||
| + | |- | ||
| + | | | ||
| + | ::Except for the even number two, which, when divided, are dissolved to units, but their parts do not admit the excess and deficiency. | ||
| + | |style="text-align:right;"|מלבד השנים מהמספר הזוג שהם כאשר נחלקו יותכו אל האחדים ולא יסבלו חלקיהם התוספת והחסרון | ||
| + | |- | ||
| + | | | ||
| + | :When it is divided into two equal or unequal parts, the even number admit only one [type] of the number, i.e. if one of its two parts is even, the other part is even, and if one of them is odd, the other part is odd. | ||
| + | |style="text-align:right;"|ולא יעבור הזוג בהחלקו בשני חלקים שוים או בלתי שוים מן אחד מן המספר ר"ל אם היה אחד משני חלקיו זוג היה האחר זוג ואם היה אחד מהם נפרד היה האחר נפרד | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *The '''odd number''' is that whose parts are unequal, regardless of how it is divided, and its parts are necessarily the even and the odd together, i.e. if one of its parts is odd the other part is even. |
| + | |style="text-align:right;"|והמספר הנפרד הוא אשר איך שתחלקהו לא ישתוו חלקיו ולא ימנעו מחלקיו הזוג והנפרד יחד ר"ל שאם היה אחד מחלקיו נפרד היה האחר זוג | ||
| + | |- | ||
| + | |Hence, it is clarified that the parts of the odds are as close to equality as possible, when there is a unit between its two parts, by which one of them exceeds the other. | ||
| + | |style="text-align:right;"|אם כן הוא מבואר שהיותר קרוב מה שיהיו חלקי הנפרד מן השווי כאשר היה בין שני חלקיו אחדות יעדיף בו אחד מהם לאחד | ||
| + | |- | ||
| + | |According to the definition that is called '''Diaulos'''[?], whose meaning is the knowledge of one of the two unknowns through the other. | ||
| + | |style="text-align:right;"|ואמנם בגדר אשר יקרא דיאלילש וענינו ידיעת אחד משני המוסכלים באחר | ||
|- | |- | ||
| | | | ||
| − | ===== The Units ===== | + | :For the odd number is that which differs from the even on its both sides by a unit, either by excess over it, or by decreasing from it. |
| + | |style="text-align:right;"|כי המספר הנפרד הוא המתחלף משני צדדיו לזוג באחדות אם בתוספת עליו ואם בחסרון ממנו | ||
| + | |- | ||
| + | | | ||
| + | :Thus, by this definition, the odd that is unknown, is unknown, until the even that is unknown, is known. | ||
| + | |style="text-align:right;"|הנה אם כן בזה הגדר לא יודע הנפרד אשר הוא מוסכל עד שיודע הזוג אשר הוא מוסכל | ||
| + | |- | ||
| + | | | ||
| + | :And the even that is unknown, is unknown, until the odd that is unknown, is known. | ||
| + | |style="text-align:right;"|ולא יודע הזוג אשר הוא מוסכל עד שיודע הנפרד אשר הוא מוסכל | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | ===== The Discussion on the Units and the Dominion of the Unity ===== | ||
| − | + | |style="text-align:right;"|<big>הדבור באחדים ושררת האחדות</big> | |
| + | |- | ||
| + | |'''Every number''' is half its two sides [adjacent numbers] when they are summed. | ||
| + | |style="text-align:right;"|כל מספר הוא חצי שתי פאותיו כאשר יקובצו | ||
| + | |- | ||
| + | | | ||
| + | *i.e. that which exceeds this number by one, and that which precedes this number by one when they are summed; | ||
| + | :<math>\scriptstyle n=\frac{1}{2}\sdot\left[\left(n+1\right)+\left(n-1\right)\right]</math> | ||
| + | |style="text-align:right;"|ר"ל מה שהוא מוסיף מאותו המספר באחד ופוחת ממנו באחד כאשר יקובצו | ||
| + | |- | ||
| + | | | ||
| + | *likewise, it is half the two sides of its sides | ||
| + | :<math>\scriptstyle n=\frac{1}{2}\sdot\left[\left(n+2\right)+\left(n-2\right)\right]</math> | ||
| + | |style="text-align:right;"|וכמו כן הוא חצי שתי פאות פאותיו | ||
| + | |- | ||
| + | | | ||
| + | *and also half of what follows, when that by which one of the two sides precedes is as that by which the other side exceeds. | ||
| + | :<math>\scriptstyle n=\frac{1}{2}\sdot\left[\left(n+m\right)+\left(n-m\right)\right]</math> | ||
| + | |style="text-align:right;"|וחצי גם כן מה שאחר זה כאשר היה מה שיחסר מאחת משתי הפיאות כשעור מה שיוסיף באחרת | ||
| + | |- | ||
| + | | | ||
| + | *and so on always, until the deficiency of the smaller side reaches the unity, beyond which it cannot pass. | ||
| + | |style="text-align:right;"|וכן יהיה תמיד עד שיגיע החסרון מהפאה הקטנה אל האחדות אשר לא יוכל לעברו | ||
| + | |- | ||
| + | |However, the '''unit''' is accompanied by the smallest number alone, which is two. | ||
| + | |style="text-align:right;"|ואמנם ה{{#annot:term|369,1686|AZ1I}}אחדות{{#annotend:AZ1I}} הנה לא ילוה לו רק הפחות במספרים אשר הוא שנים | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :As it is not a number that has two sides, it is half its one side, i.e. half of the two. |
| + | :<math>\scriptstyle{\color{blue}{1=\frac{1}{2}\sdot2}}</math> | ||
| + | |style="text-align:right;"|ולפי שאינו מספר שיהיו לו שתי פיאות היה חצי פאתו האחת ר"ל חצי השנים | ||
|- | |- | ||
| | | | ||
| − | ===== Even Number ===== | + | :Since the production of the two is from its doubling |
| + | :<math>\scriptstyle{\color{blue}{2=2\sdot1}}</math> | ||
| + | |style="text-align:right;"|אחר שתולדת השנים מכפלו | ||
| + | |- | ||
| + | |Hence, it is already clarified that the unit is the cause of number in its growth. | ||
| + | |style="text-align:right;"|הנה כבר התבאר שהאחדות עלת המספר בצמיחתו | ||
| + | |- | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ===== The Discussion on the Categories of the Even Number and the Property of the Even-Times-Even Number ===== | ||
| − | + | |style="text-align:right;"|<big>הדבור בחלקי הזוג וסגולתו זוג הזוג</big> | |
| + | |- | ||
| + | |The even number is divided into three categories: | ||
| + | |style="text-align:right;"|המספר הזוג יחלק לשלשה חלקים | ||
| + | |- | ||
| + | | | ||
| + | :1) even-times-even | ||
| + | |style="text-align:right;"|אחד מהם זוג הזוג | ||
|- | |- | ||
| | | | ||
| − | ====== Even-Times Even ====== | + | :2) even-times-odd |
| + | |style="text-align:right;"|והשני זוג הנפרד | ||
| + | |- | ||
| + | | | ||
| + | :3) even-times-even-times-odd | ||
| + | |style="text-align:right;"|והשלישי זוג הזוג והנפרד | ||
| + | |- | ||
| + | | | ||
| + | *The first two types, which are the even-times-even and the even-times-odd, are distinguished by the difference between the even and the odd by definition. | ||
| + | |style="text-align:right;"|הנה אם כן שני החלקים הראשונים אשר הם זוג הזוג וזוג הנפרד נבדלים להבדל הזוג והנפרד בגדר | ||
| + | |- | ||
| + | | | ||
| + | *The third type, which is the even-times-even-times-odd, is as a mean between the two extremes, since it assumes the name that consists of the names of both types. | ||
| + | |style="text-align:right;"|והחלק השלישי אשר הוא זוג הזוג והנפרד כאמצעי משני הקצוות ללקחו השם מורכב משמות שני החלקים | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | ====== Even-Times-Even Number ====== | ||
| − | + | |style="text-align:right;"|<big>הנה זוג הזוג</big> | |
| + | |- | ||
| + | | | ||
| + | *It consists of doubling the unit. | ||
| + | |style="text-align:right;"|הוא מורכב מהכפלת האחד | ||
| + | |- | ||
| + | | | ||
| + | *Therefore it is divisible into two equal parts, which are its halves, | ||
| + | |style="text-align:right;"|אם כן הוא אשר איפשר שיחלק בשני חלקים שוים הם חצאיו | ||
| + | |- | ||
| + | | | ||
| + | :each of its halves [is divisible] into two halves, | ||
| + | |style="text-align:right;"|וכל חצי מהם לשני חצאים | ||
| + | |- | ||
| + | | | ||
| + | :and also the halves of its halves, | ||
| + | |style="text-align:right;"|וכן חצאי חצאיו | ||
| + | |- | ||
| + | | | ||
| + | :until reaching the one, since it is generated from its doubling, as explained previously. | ||
| + | |style="text-align:right;"|עד שיגיע אל האחד לפי שהוא מהכפלתו כמו שבארנו לפנים | ||
| + | |- | ||
| + | | | ||
| + | *Hence, it is already clarified that each half of the even-times-even is an even-times-even, | ||
| + | |style="text-align:right;"|הנה אם כן כבר התבאר שכל אחד מחצי זוג הזוג זוג הזוג | ||
| + | |- | ||
| + | | | ||
| + | :and so are the halves of its halves, | ||
| + | |style="text-align:right;"|וכן חצי חצייו | ||
| + | |- | ||
| + | | | ||
| + | :since they all have halves and their halves have halves and so on until reaching the unit. | ||
| + | |style="text-align:right;"|לפי שכל אלו להם חציים ולחצייהם חציים עד שיגיעו אל האחדות | ||
| + | |- | ||
| + | | | ||
| + | *Furthermore, the names of the parts of an even-times-even number are always derived from and even number, which is an even-times-even number, and their values are also even-times-even numbers. | ||
| + | |style="text-align:right;"|ועוד כי חלקי זוג הזוג נמצאים לעולם נגזרי השם מזוג הוא זוג הזוג והגעתם ג"כ זוג הזוג | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\frac{1}{8}\sdot64=8}}</math> → 8 is even-times-even |
| + | |style="text-align:right;"|כמו שמינית ס"ד אשר הוא נגזר משמנה אשר הוא זוג הזוג והגעתו שמנה והוא זוג הזוג | ||
|- | |- | ||
| | | | ||
| − | ====== Even-Times Odd ====== | + | ::<math>\scriptstyle{\color{blue}{\frac{1}{4}\sdot64=16}}</math> → 4 and 16 are even-times-even |
| + | |style="text-align:right;"|גם כן כמו רביעיתם הנגזר בארבעה והוא זוג הזוג והגעתו י"ו | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{1}{2}\sdot64=32}}</math> → 2 and 32 are even-times-even | ||
| + | |style="text-align:right;"|וכן חצים והוא נגזר משנים והוא זוג הזוג והגעתו ל"ב והוא זוג הזוג | ||
| + | |- | ||
| + | | | ||
| + | *An even-times-even number has no part, the name of which is derived from an odd number [= indivisible by an odd number] | ||
| + | |style="text-align:right;"|ולא ימצא לזוג הזוג חלק נגזר השם ממספר נפרד | ||
| + | |- | ||
| + | | | ||
| + | ::such as <math>\scriptstyle{\color{blue}{\frac{1}{9}}}</math> which is derived from 9 | ||
| + | |style="text-align:right;"|כמו התשיעית הנגזר מתשעה | ||
| + | |- | ||
| + | | | ||
| + | ::and <math>\scriptstyle{\color{blue}{\frac{1}{3}}}</math> which is derived from 3 | ||
| + | |style="text-align:right;"|והשלישית הנגזר מג‫' | ||
| + | |- | ||
| + | | | ||
| + | *It is thought that this number is called even-times-even, because its parts are derived from the names of even numbers, and their values are also even numbers. | ||
| + | |style="text-align:right;"|וכבר יחשב שזה המספר אמנם נקרא זוג הזוג לפי שחלקיו נגזרי השמות ממספרים זוגות והגעתם זוגות גם כן | ||
| + | |- | ||
| + | | | ||
| + | *We find amoung the properties of the even-times-even number, that since the names of its parts are derived from the even-times-even numbers, their values are also even-times-even parts of that number, of which they are parts. | ||
| + | |style="text-align:right;"|ונמצא מסגולת זוג הזוג שחלקיו אחר שהם נגזרי השמות ממספרי זוג הזוגות שאותם הזוגות גם כן הם הגעות חלקי זוגות זוגות מן המספר אשר הם לו חלקים | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{1}{2}\sdot64=32\longleftrightarrow\frac{1}{32}\sdot64=2}}</math> | ||
| + | |style="text-align:right;"|כמו החצי הנגזר מב' והגעתו ל"ב מס"ד אשר החלק מל"ב מהם ב‫' | ||
| + | |- | ||
| + | | | ||
| + | *When the terms of the even-times-even numbers are set by doubling, from the one, according to this order: 1; 2; 4; 8; 16; 32; 64; 128, | ||
| + | |style="text-align:right;"|וכאשר הנחנו מדרגות מספרי זוג הזוג בהכפלה מהאחד כפי זה הסדר א' ב' ד' ח' י"ו ל"ב ס"ד קכ"ח | ||
| + | |- | ||
| + | | | ||
| + | ::there are eight terms - the first term is 1; the eighth term is 128 | ||
| + | |style="text-align:right;"|היו שמנה מדרגות הראשונה א' והשמינית קכ"ח | ||
| + | |- | ||
| + | | | ||
| + | ::and they have two mean terms, which are 8 and 16. | ||
| + | |style="text-align:right;"|והיו להם שני {{#annot:term|2121,1741|anLc}}אמצעיים{{#annotend:anLc}} והם ח' וי"ו | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{1}{16}\sdot128=8}}</math> | ||
| + | |style="text-align:right;"|והיה החלק מי"ו מקכ"ח ח‫' | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{1}{8}\sdot128=16}}</math> | ||
| + | |style="text-align:right;"|ושמינית קכ"ח י"ו | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{1}{32}\sdot128=4}}</math> | ||
| + | |style="text-align:right;"|וכמו כן החלק מל"ב מהם ד‫' | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{1}{4}\sdot128=32}}</math> | ||
| + | |style="text-align:right;"|ורביע קכ"ח ל"ב | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{1}{64}\sdot128=2}}</math> | ||
| + | |style="text-align:right;"|וכמו החלק מס"ד מהם ב‫' | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{1}{2}\sdot128=64}}</math> | ||
| + | |style="text-align:right;"|וחציים ס"ד | ||
| + | |- | ||
| + | | | ||
| + | ::likewise, 128 is one time in the unit, as the one is 128 times in [128]. | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{1}{1}\sdot128=128}}</math> | ||
| + | |style="text-align:right;"|וכמו כן היה באחדות פעם אחת קכ"ח כמו שהאחד בם קכ"ח פעם | ||
| + | |- | ||
| + | | | ||
| + | *It is already clarified that the names of the parts of the even-times-even numbers are derived from their values that are contained in them in accordance with the doubling, as described previously. | ||
| + | |style="text-align:right;"|הנה כבר התבאר במספרי זוג הזוג חלקיהם נגזרי השמות ממה שבתוכם מהגעתם כפי ההכפלה אשר תארנוה לפנים | ||
| + | |- | ||
| + | | | ||
| + | *Likewise, if one term is subtracted from their terms, so that the number of their terms will be an odd number: | ||
| + | |style="text-align:right;"|וכמו כן גם כן אם יושלך ממדרגותיהם מדרגה עד שיהיה מספר מדרגותיהם נפרד | ||
| + | |- | ||
| + | | | ||
| + | ::The greatest extreme of them will be 64. | ||
| + | |style="text-align:right;"|ויהיה הקצה הגדול מהם ס"ד | ||
| + | |- | ||
| + | | | ||
| + | ::You find that nothing is detracted from what was described: | ||
| + | |style="text-align:right;"|תמצא שלא יבצר דבר ממה שתארנו | ||
| + | |- | ||
| + | | | ||
| + | ::the mean term: <math>\scriptstyle{\color{blue}{\frac{1}{8}\sdot64=8}}</math> | ||
| + | |style="text-align:right;"|ויהיה שמיניתו ח' אשר הוא ה{{#annot:term|2121,1741|pd2u}}אמצעי{{#annotend:pd2u}} | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{1}{16}\sdot64=4}}</math> | ||
| + | |style="text-align:right;"|וחלק מי"ו מהם ד‫' | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{1}{4}\sdot64=16}}</math> | ||
| + | |style="text-align:right;"|ורביעיתם י"ו | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{1}{32}\sdot64=2}}</math> | ||
| + | |style="text-align:right;"|והחלק מל"ב מהם ב‫' | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{1}{2}\sdot64=32}}</math> | ||
| + | |style="text-align:right;"|וחציים ל"ב | ||
| + | |- | ||
| + | | | ||
| + | ::64 is one time in the unit, as the one is 64 times in [64]. | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{1}{1}\sdot64=64}}</math> | ||
| + | |style="text-align:right;"|וס"ד באחד ס"ד פעם כמו שהאחד בו ס"ד פעם | ||
| + | |- | ||
| + | | | ||
| + | *It is also necessary for the terms of the even-times-even number, that when they are placed in the order that was given as an example, and the number of terms is even, they will have two means. | ||
| + | |style="text-align:right;"|ועוד יתחייב למדרגות זוג הזוג כאשר יונחו על הסדר אשר המשלנו והיו המדרגות זוג ויחויב שיהיו להם שני אמצעיים | ||
| + | |- | ||
| + | | | ||
| + | :the product of one of the two means by the other is equal to the product of the two extremes one by the other, | ||
| + | :<math>\scriptstyle2^{\frac{2n-2}{2}}\sdot2^{\frac{2n}{2}}=1\sdot2^{2n-1}</math> | ||
| + | |style="text-align:right;"|יהיה מה שיתקבץ מהכאת אחד משני האמצעיים באחר שוה למה שיתקבץ מהכאת שני הקצוות אחד מהם באחר | ||
| + | |- | ||
| + | | | ||
| + | :and [to the product of the terms] that follow the extremes one by the other, | ||
| + | :<math>\scriptstyle2^{\frac{2n-2}{2}}\sdot2^{\frac{2n}{2}}=2\sdot2^{2n-2}</math> | ||
| + | |style="text-align:right;"|וכן מה שילווה לשני הקצוות אחד מהם באחר | ||
| + | |- | ||
| + | | | ||
| + | :and [to the product of the terms] that follow what follows [the extremes one by the other], | ||
| + | :<math>\scriptstyle2^{\frac{2n-2}{2}}\sdot2^{\frac{2n}{2}}=2^2\sdot2^{2n-3}</math> | ||
| + | |style="text-align:right;"|ומה שילוה לנלוה | ||
| + | |- | ||
| + | | | ||
| + | :until reaching the terms that follow the means. | ||
| + | :<math>\scriptstyle2^{\frac{2n-2}{2}}\sdot2^{\frac{2n}{2}}=2^{\frac{2n-4}{2}}\sdot2^{\frac{2n+2}{2}}</math> | ||
| + | |style="text-align:right;"|עד שיגיע לנלוים לשני האמצעיים | ||
| + | |- | ||
| + | | | ||
| + | :Examine this rule in the terms that were counted, whose number is even, whose beginning is 1 and end is 128, and you will find them as was said. | ||
| + | |style="text-align:right;"|ותבחן הקש זה במדרגות אשר ספרנום שיהיה מספרם זוג והם אשר תחלתם אחד וסופם קכ"ח ותמצא כמו שאמרנו | ||
| + | |- | ||
| + | | | ||
| + | *When the number of terms is odd, they have one mean. | ||
| + | |style="text-align:right;"|וכאשר היה מספר המדרגות נפרד היה להם אמצעי אחד | ||
| + | |- | ||
| + | | | ||
| + | :the product of the mean by itself is equal to the product of the two extremes one by the other, | ||
| + | :<math>\scriptstyle\left(2^n\right)^2=1\sdot2^{2n}</math> | ||
| + | |style="text-align:right;"|ויהיה מוכה האמצעי בעצמו כהכאת שני הקצוות אחד מהם באחר | ||
| + | |- | ||
| + | | | ||
| + | :and [to the product of the terms] that follow [the extremes], as explained. | ||
| + | :<math>\scriptstyle\left(2^n\right)^2=2\sdot2^{2n-1}</math> | ||
| + | |style="text-align:right;"|וכן מה שימשך להם כמו שבארנו | ||
| + | |- | ||
| + | | | ||
| + | *It is necessary for the terms of the even-times-even numbers that when they are summed from the one, the sum of all of them is one less than the greater extreme. | ||
| + | :<math>\scriptstyle\sum_{i=1}^{n} 2^{i-1}=2^n-1</math> | ||
| + | |style="text-align:right;"|וכבר יתחייב למדרגת זוג הזוג שיהיו כאשר נכללו מן האחד היה הגעת כלם פחות מהקצה הגדול באחד | ||
| + | |- | ||
| + | | | ||
| + | *If the greater extreme is taken with them, the total is one less than double the greater extreme. | ||
| + | :<math>\scriptstyle\sum_{i=1}^{n+1} 2^{i-1}=2\sdot2^n-1</math> | ||
| + | |style="text-align:right;"|ואם לוקח עמם הקצה הגדול היה כל זה פחות מכפל הקצה הגדול באחד | ||
| + | |- | ||
| + | | | ||
| + | :This is necessary for the terms, be they few or many. | ||
| + | |style="text-align:right;"|וזה יתחייב למדרגות ימעטו או ירבו | ||
| + | |- | ||
| + | | | ||
| + | *It is clarified from this that the terms of even-times-even numbers, when they are summed from one, their sum is an odd number, as they are one less than the greater extreme, which is always an even number. | ||
| + | |style="text-align:right;"|ומבואר מזה שמדרגות זוג הזוג כשנכללו מן האחד היה הגעתם נפרד לפי שהם פחות מהקצה הגדול אשר הוא לעולם זוג באחד | ||
| + | |- | ||
| + | | | ||
| + | ::One less than an even number is always an odd number: | ||
| + | ::2n-1 | ||
| + | |style="text-align:right;"|והחסר מהזוג אחד הוא הנפרד | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ====== The Discussion on the Even-Times-Odd ====== | ||
| − | + | |style="text-align:right;"|<big>הדבור בזוג הנפרד</big> | |
| + | |- | ||
| + | |The number that is an even-times-odd number is divided into two parts of similar units, by the nature of its type, as it is an even number, but it stops there and none of its halves can be divided again into halves. | ||
| + | |style="text-align:right;"|המספר אשר הוא זוג הנפרד יחלק לשני חלקים דומי האחדים למה שתקנהו מטבע סוגו אחר שהוא זוג ושם יעמוד ולא יתחלק פעם שנית אחד מחלקיו בשני חצאים | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *Hence, the even-times-odd number is doubling the odd number once. |
| + | :<math>\scriptstyle2\sdot\left(2n-1\right)</math> | ||
| + | |style="text-align:right;"|הנה אם כן זוג הנפרד הוא הכפלת הנפרד פעם אחת | ||
| + | |- | ||
| + | | | ||
| + | ::examples: 6; 10; 14; 18; 22; 26 | ||
| + | |style="text-align:right;"|כמו ששה ועשרה וארבעה עשר וי"ח וכ"ב וכ"ו | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{6=2\sdot3}}</math> | ||
| + | |style="text-align:right;"|כי ששה מכפל ג‫' | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{10=2\sdot5}}</math> | ||
| + | |style="text-align:right;"|ועשרה מכפל ה‫' | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{14=2\sdot7}}</math> | ||
| + | |style="text-align:right;"|וי"ד מכפל ז‫' | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{18=2\sdot9}}</math> | ||
| + | |style="text-align:right;"|וי"ח מכפל ט‫' | ||
| + | |- | ||
| + | !<span style=color:red>[The production of the even-times-odd numbers:]</span> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :*When the natural odd numbers are ordered, i.e. by the natural successive order, starting from the beginning of the odd numbers, which is 3. | ||
| + | |style="text-align:right;"|הנה אם כן כשיסודרו הנפרדים הטבעיים ר"ל על משך סדר הטבע ויתחילו מתחלת הנפרדים אשר הוא ג‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Such as: 3; 5; 7; 9; 11; 13 | ||
| + | |style="text-align:right;"|כמו ג' ה' ז' ט' י"א י"ג | ||
| + | |- | ||
| + | | | ||
| + | ::The excess of each odd number over the preceding odd number is 2. | ||
| + | |style="text-align:right;"|והיה תוספת הנפרד על הנפרד אשר לפניו מהם שנים שנים | ||
| + | |- | ||
| + | | | ||
| + | :*Then these odd numbers are doubled and arranged according to their succession. | ||
| + | |style="text-align:right;"|עוד תכפול אלו הנפרדים על המשכם ותסדרם | ||
| + | |- | ||
| + | | | ||
| + | ::Such as: 6; 10; 14; 18 | ||
| + | |style="text-align:right;"|כמו ו' י' י"ד י"ח | ||
| + | |- | ||
| + | | | ||
| + | ::Each exceeds the preceding number by 4. | ||
| + | |style="text-align:right;"|תוסיף כל פעם על אשר לפניו ד"ד | ||
| + | |- | ||
| + | | | ||
| + | :::Since the natural odd numbers, which form these even numbers by their doubling, are added two by two, | ||
| + | |style="text-align:right;"|לפי שהנפרדים הטבעיים אשר חדשו אלו הזוגות מכפלם היו נוספים שנים שנים | ||
| + | |- | ||
| + | | | ||
| + | :::and when they are doubled, the excess is doubled, so it is 4 by 4, [<math>\scriptstyle{\color{blue}{2\times2=4}}</math>], as illustrated in their terms. | ||
| + | |style="text-align:right;"|וכאשר נכפלו יכפל התוספת והיו ד"ד כמו שהמשלנוהו ממדרגותיהם | ||
| + | |- | ||
| + | | | ||
| + | :*It is clear that they are formed in their natural succession by doubling once the natural odd numbers. | ||
| + | |style="text-align:right;"|ומבואר שהם אמנם יתחדשו על משך טבעם מהכפלת הנפרדים הטבעיים פעם אחת | ||
| + | |- | ||
| + | | | ||
| + | *It is necessary for the even-times-odd number that the genus of the value of each of its parts differs from the genus of the names of their derivation. | ||
| + | |style="text-align:right;"|וכבר יתחייב לזוג הנפרד שיהיה כל אחד מחלקיו מתחלף ההגעה לשמות גזרתו בסוג | ||
| + | |- | ||
| + | | | ||
| + | :I.e., if the name of the part is derived from an odd number - its value is an even number, | ||
| + | |style="text-align:right;"|ר"ל שאם היה החלק נגזר השם ממספר נפרד היה הגעתו זוג | ||
| + | |- | ||
| + | | | ||
| + | :and if the name of the part is derived from an even number - its value is an odd number. | ||
| + | |style="text-align:right;"|ואם היה החלק נגזר השם ממספר זוג היה הגעתו נפרד | ||
| + | |- | ||
| + | | | ||
| + | ::Such as six of the even-times-odd [type] | ||
| + | |style="text-align:right;"|כמו ששה מזוג הנפרד | ||
| + | |- | ||
| + | | | ||
| + | ::for its third, which is derived from 3 that is odd, is 2: <math>\scriptstyle{\color{blue}{\frac{1}{3}\sdot6=2}}</math> | ||
| + | |style="text-align:right;"|כי שלישיתו הנגזר מג' והוא נפרד ב‫' | ||
| + | |- | ||
| + | | | ||
| + | ::and its half, which is derived from 2 that is even, is 3 that is odd: <math>\scriptstyle{\color{blue}{\frac{1}{2}\sdot6=3}}</math> | ||
| + | |style="text-align:right;"|וחציו הנגזר מב' והוא זוג ג' והוא נפרד | ||
| + | |- | ||
| + | | | ||
| + | :Thus, it is clear that the even-times-even number differs from the even-times-odd number, since the parts of the even-times-even number are named after numbers of the same genus as their values. | ||
| + | |style="text-align:right;"|אם כן כבר התבאר שזוג הזוג מתחלף לזוג הנפרד לפי שזוג הזוג חלקיו נגזרי השמות מסוג הגעתם | ||
| + | |- | ||
| + | | | ||
| + | ::Such as 8, whose quarter is 2: <math>\scriptstyle{\color{blue}{\frac{1}{4}\sdot8=2}}</math>, and whose half is 4: <math>\scriptstyle{\color{blue}{\frac{1}{2}\sdot8=4}}</math> | ||
| + | |style="text-align:right;"|כמו ח' אשר רביעיתם ב' וחציים ד‫' | ||
| + | |- | ||
| + | | | ||
| + | :It is thought that because of this, this type is called even-times-odd, since the genus of the names of their parts differs from the genus of their value. | ||
| + | |style="text-align:right;"|וכבר יחשב שלזה נקרא זה המין זוג הנפרד אחר שהיו חלקיו מתחלפים בשם ובהגעה בסוג | ||
| + | |- | ||
| + | | | ||
| + | *It is necessary for the even-times-odd numbers that none of them will have a third [= divisible by 3], except for those that will be described: | ||
| + | |style="text-align:right;"|וכבר יתחייב לזוג הנפרד שלא יהיה לדבר ממנו שליש אלא למה שאתאר | ||
| + | |- | ||
| + | | | ||
| + | :*Setting the terms of the even-times-odd numbers starting from 6, according to the example described previously, which are: 6; 10; 14; 18; 22; 26; 30; 34; 38; 42; 46; 50; 54 | ||
| + | |style="text-align:right;"|וזה שתניח מדרגות זוג הנפרד אשר התחלתם ו' על הדמיון המתואר קודם זה<br> | ||
| + | והם ו' י' י"ד י"ח כ"ב כ"ו ל' ל"ד ל"ח מ"ב מ"ו נ' נ"ד | ||
| + | |- | ||
| + | | | ||
| + | ::Skipping over three terms from the beginning of the terms - the fourth term has a third: <math>\scriptstyle{\color{blue}{\frac{1}{3}\sdot18=6}}</math> | ||
| + | |style="text-align:right;"|עוד נעבור שלשה מדרגות מתחלת המדרגות הנה הרביעית יש לה שליש והיא י"ח ושלישיתה ו‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Proceding three terms further from 18 - the fourth term has a third: <math>\scriptstyle{\color{blue}{\frac{1}{3}\sdot30=10}}</math> | ||
| + | |style="text-align:right;"|עוד תתרחק מי"ח שלשה מדרגות והרביעית יש שליש והיא ל' ושלישיתה י‫' | ||
| + | |- | ||
| + | | | ||
| + | ::And so on always, the even-times-odd numbers that have a third [= divisible by 3] will not escape from you. | ||
| + | |style="text-align:right;"|וכן תמיד ולא ימלטו ממך מזוג הנפרד מה שיש לו שליש | ||
| + | |- | ||
| + | !<span style=color:red>[The production of the even-times-odd numbers from the sequence of even numbers that are divisible by 3:]</span> | ||
| + | | | ||
| + | |- | ||
| + | |'''Abū Yusūf''' said: | ||
| + | |style="text-align:right;"|<big>אמר אבו יוסף</big> | ||
| + | |- | ||
| + | | | ||
| + | :*When taking the even numbers that have a third [= divisible by 3] from their beginning and arranging them successively, | ||
| + | |style="text-align:right;"|ואתה כאשר תקח הזוגות אשר להם שליש מתחלתם ותסדרם ימשכו קצתם לקצת | ||
| + | |- | ||
| + | | | ||
| + | ::such as: 18; 30; 42; 54; 66 | ||
| + | |style="text-align:right;"|כמו י"ח ל' ומ"ב ונ"ד וס"ו | ||
| + | |- | ||
| + | | | ||
| + | :*Then taking a third of each of them by succession and arranging them successively, | ||
| + | |style="text-align:right;"|ותקח שליש כל אחד מהם על המשך ותסדרם ימשכו קצתם לקצת | ||
| + | |- | ||
| + | | | ||
| + | ::such as: 6; 10; 14; 18; 22 | ||
| + | |style="text-align:right;"|כמו ו' י' י"ד י"ח כ"ב | ||
| + | |- | ||
| + | | | ||
| + | :*You find their thirds by their successive order are the terms of the even-times-odd number from their beginning by their order, none of them is missing. | ||
| + | |style="text-align:right;"|תמצא שלשיותיהם על משך סדרם הם מדרגות זוג הנפרד מתחלתם על סדרם לא יבצר מהם דבר | ||
| + | |- | ||
| + | | | ||
| + | *It is established concerning the even-times-odd number, that when the number of its terms is even, having two means, the sum of the two means is equal to the sum of the two extremes, and to the sum of the terms that follow the extremes, and to the sum of the terms that follow the terms that follow [the extremes]. | ||
| + | :<math>\scriptstyle\left[2\sdot\left(2n+1\right)\right]+\left[2\sdot\left[\left[2\sdot\left(n+1\right)\right]+1\right]\right]=\left[2\sdot\left(2+1\right)\right]+\left[2\sdot\left[\left(2\sdot2n\right)+1\right]\right]</math> | ||
| + | |style="text-align:right;"|וכבר ישיג לזוג הנפרד שמדרגותיו כאשר היה מספרם זוג והיו להם שני אמצעיים היו האמצעים כמו שני הקצוות מקובצים וכמו אשר ילוה לשני הקצוות ואשר ילוה לנלוה | ||
| + | |- | ||
| + | | | ||
| + | :As explained previously concerning the terms of the even-times-even numbers, except that the issue there was multiplication, and here it is addition. | ||
| + | |style="text-align:right;"|כמו שבארנו לפנים במדרגות זוג הזוג אלא שהענין יהיה שם בהכאה ובכאן יהיה בחבור | ||
| + | |- | ||
| + | | | ||
| + | *If the number of the terms is an odd number - they have only one mean. | ||
| + | |style="text-align:right;"|ואם היו מספר המדרגות נפרד היה להם אמצעי אחד לבד | ||
| + | |- | ||
| + | | | ||
| + | :Double the one mean of the terms of an even-times-even number is equal to the sum of the two extremes, and to the sum of the terms that follow the extremes, and to the sum of the terms that follow the terms that follow [the extremes]. | ||
| + | |style="text-align:right;"|ותהיה הכפלת האמצעי האחד ממדרגות זוג הנפרד כמו מקובץ שני הקצוות וכן מה שימשך לשני הקצוות ומה שימשך לנמשך אליהם | ||
| + | |- | ||
| + | |Hence, the difference of the even-times-even numbers and the even-times-odd numbers is already clear with regard to difference between the issue of the product and the issue of the sum of the two extremes, the single mean, or the two means. | ||
| + | |style="text-align:right;"|הנה כבר התבאר הנה חלוף זוג הזוג וזוג הנפרד בהתחלף ענין ההכאה וענין החבור בשני הקצוות והאמצעי ושני האמצעיים | ||
| + | |- | ||
| + | |Furthermore, the even-times-even number and the even-times-odd number differ from each other also in that the even-times-odd number alone is divisible [= its other divisors are indivisible by 2], while the only indivisible part of the even-times-even number is the smallest part, i.e. 1 [= its other divisors are divisible by 2] | ||
| + | |style="text-align:right;"|וכבר יתחלף זוג הזוג לזוג הנפרד גם כן כאשר המתחלק מזוג הנפרד קצהו הגדול לבד ושהבלתי מתחלק מזוג הזוג קצהו הקטן לבד אשר הוא האחד | ||
|- | |- | ||
| | | | ||
| − | |||
| − | + | ====== The Discussion on the Quality of the Even-Times-Even-Times-Odd ====== | |
| + | |||
| + | |style="text-align:right;"|<big>הדבור בתאר זוג הזוג והנפרד</big> | ||
| + | |- | ||
| + | |The even-times-even-times-odd number is the third species of even numbers | ||
| + | |style="text-align:right;"|אמנם המספר אשר הוא זוג הזוג והנפרד והוא המין השלישי ממיני הזוג | ||
| + | |- | ||
| + | |It is as a mean between the [other types of even numbers] and consists of them, since it adopts from each of them its property | ||
| + | |style="text-align:right;"|כאמצעי להם ומורכב מהם ללוקחו מכל אחד כמו סגלתו | ||
| + | |- | ||
| + | | | ||
| + | *The similarity it adopts from the even-times-even number: it is divisible into two equal parts by the nature granted from its genus. | ||
| + | |style="text-align:right;"|אמנם מה שלקח מדמיון זוג הזוג שהוא יחלק לשני חלקים מתמשלי האחדים בטבע אשר הקנהו סוגו | ||
| + | |- | ||
| + | | | ||
| + | :Each of its two parts is divisible into two equal parts by the nature granted to it necessarily. | ||
| + | |style="text-align:right;"|עוד יחלק כל אחד משני חלקיו שנית בשני חלקים מתמשלי האחדים בטבע אשר הקנהו בהכרח | ||
| + | |- | ||
| + | | | ||
| + | :Its parts and the parts of its parts may be divided into two equal parts as well repeatedly, but the division does not reach the unit. | ||
| + | |style="text-align:right;"|ואולי יחלקו חלקיו וחלקי חלקיו פעמים רבות אלא שלא תגיע בו החלוקה אל האחד | ||
| + | |- | ||
| + | | | ||
| + | :Hence, as it can be divided into two equal parts more than once it is similar to the even-times-even number and differs from the even-times-odd number. | ||
| + | |style="text-align:right;"|הנה במה שיחלק לשני חלקים מתמשלי האחדים יותר מפעם אחת ידמה לזוג הזוג ויתחלף לזוג הנפרד | ||
| + | |- | ||
| + | | | ||
| + | :As its halving does not end with the unit it is similar to the even-times-odd number and differs from an even-times-even number. | ||
| + | |style="text-align:right;"|ובאשר לא יכלה בחצוי אל האחד ידמה לזוג הנפרד ויתחלף לזוג הזוג | ||
| + | |- | ||
| + | |By every property, by which it is similar to one of the two species, it differs from the other species. | ||
| + | |style="text-align:right;"|הנה כל מה שידמה בו אחד משני המינים יתחלף באותו הענין למין האחר | ||
| + | |- | ||
| + | | | ||
| + | ::This can be seen in the even-times-even-times-odd numbers, such as: 12; 20; 24. | ||
| + | |style="text-align:right;"|ותבחן זה במספרי זוג הזוג והנפרד כמו י"ב וכ' וכ"ד ותמצאהו כן | ||
| + | |- | ||
| + | |The even-times-even-times-odd number has properties that the even-times-even and the even-times-odd numbers do not have; and it has properties that are shared by them as well. | ||
| + | |style="text-align:right;"|וכבר ישיג מספר זוג הזוג והנפרד שימצאו לו הסגלות אשר נאמרו שלא ימצאו לזוג הזוג ולא לזוג הנפרד ושימצאו לו ג"כ אותם הסגלות אשר ימצאו אליהם | ||
| + | |- | ||
| + | | | ||
| + | *[A property that the even-times-even-times-odd number] has while the other do not: | ||
| + | |style="text-align:right;"|אמנם אשר ישיגהו ממה שלא ישיגם | ||
| + | |- | ||
| + | | | ||
| + | :Only the greatest part of the even-times-odd number is divisible [= its other divisors are indivisible by 2]. | ||
| + | |style="text-align:right;"|מזה שהמתחלק מזוג הנפרד קצהו הגדול לבד | ||
| + | |- | ||
| + | | | ||
| + | :The only indivisible part of the even-times-even number is the smallest part [= i.e. 1; its other divisors are divisible by 2]<br> | ||
| + | |style="text-align:right;"|והבלתי מתחלק מזוג הזוג קצהו הקטן לבד | ||
| + | |- | ||
| + | | | ||
| + | :Yet, for the even-times-even-times-odd number, its the greatest part is not the only divisible part, and its smallest part is not the only indivisible part, but it has divisible parts other than its greatest part, and it has necessarily indivisible parts other than the one. | ||
| + | |style="text-align:right;"|אבל זוג הזוג והנפרד אין המתחלק לבד קצהו הגדול ולא הבלתי מתחלק ממנו קצהו הקטן לבד אבל כבר ימצאו לו בלתי קצהו הגדול חלקים יקבלו ההחלק גם כן וימצאו לו בהכרח קודם שיכלה בו ההחלק אל האחד חלק לא יקבל חלוקה | ||
| + | |- | ||
| + | | | ||
| + | *[A property that the even-times-even-times-odd number] shares with the others: | ||
| + | |style="text-align:right;"|ואמנם מה שישיגהו ממה שישיגם | ||
| + | |- | ||
| + | | | ||
| + | :It has parts that are denominated by a number of the same species as the species of their value - by this it is similar to the even-times-even number. | ||
| + | |style="text-align:right;"|הוא שבו חלקים נגזרי השמות ממספר מין הגעתם דומה בזה לזוג הזוג | ||
| + | |- | ||
| + | | | ||
| + | :It has parts whose derivation differs from their value in their genera - by this it is similar to the even-times-odd number. | ||
| + | |style="text-align:right;"|ובו חלקים מתחלפים הגזרה במין להגעתם ידמה בזה לזוג הנפרד | ||
| + | |- | ||
| + | |Examine this and you will find it so. | ||
| + | |style="text-align:right;"|ותבחן זה ותמצאהו כן | ||
| + | |- | ||
| + | !<span style=color:red>[The production of the even-times-even-times-odd numbers:]</span> | ||
| + | | | ||
| + | |- | ||
| + | |How this species is produced and grows: | ||
| + | |style="text-align:right;"|ואמנם איך יתילד זה המין ואיך יצמח | ||
| + | |- | ||
| + | |Since it shares similarities with the even-times-even number and the even-times-odd number, it consists of what they both consist of | ||
| + | |style="text-align:right;"|הנה למה שהיה לוקח הדמוי לזוג הזוג ולזוג הנפרד יודע שהוא יורכב ממה שהורכבו ממנו יחד | ||
| + | |- | ||
| + | | | ||
| + | *The even-times-odd numbers are generated from doubling the natural odd numbers. | ||
| + | |style="text-align:right;"|הנה המספר אשר הוא זוג הנפרד אמנם יתילד מהכפלת הנפרדים הטבעיים | ||
| + | |- | ||
| + | | | ||
| + | *The even-times-even numbers are generated from doubling the unit. | ||
| + | |style="text-align:right;"|והמספר אשר הוא זוג הזוג אמנם יתילד מהכפלת האחדים | ||
| + | |- | ||
| + | |Generating the third type, which is the even-times-even-times-odd numbers: | ||
| + | |style="text-align:right;"|הנה כאשר תרצה להוליד זה המין השלישי אשר הוא זוג הזוג והנפרד | ||
| + | |- | ||
| + | | | ||
| + | :*Arranging the natural odd numbers, starting from 3, succeeding one by one in a line lengthwise [column 2]. | ||
| + | |style="text-align:right;"|תסדר הנפרדים הטבעיים אשר התחלתם שלשה ימשכו קצתם לקצת בטור הארוך | ||
| + | |- | ||
| + | | | ||
| + | :*Arranging the even-times-even terms, doubled from 4, succeeding one by one in a line breadthwise [row 1]. | ||
| + | |style="text-align:right;"|עוד תסדר מדרגות זוג הזוג אשר הכפלתם מד' ר"ל בזה הצד לפי שאחר הנפרד הראשון מהנפרדים הטבעיים התחלת זוג הזוג ימשכו קצתם לקצת בטור הרחב | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*Multiplying the first term of whichever line one wishes by all the terms of the other line one by one, then placing all products correspondingly in a third line [column 3]. |
| + | |style="text-align:right;"|עוד תתחיל ותכה המדרגה הראשונה מאי זה משני הטורים שתרצה בכל מדרגות הטור האחר אחד אחד<br> | ||
| + | עוד תעמיד כל מה שיצא לך על מדרגותיו בטור שלישי | ||
|- | |- | ||
| | | | ||
| + | :*Returning to the term succeeding the term that was multiplied by all the terms of the other line, applying on it what was done with the preceeding: multiplying it by all the terms of the other line and placing the products also correspondingly in a fourth line [column 4]. | ||
| + | |style="text-align:right;"|עוד אחר זה תשוב אל המדרגה הנמשכת למדרגה אשר הכית בכל מדרגות הטור האחר ותעשה בם גם כן כמו שעשית באשר לפניה ותכה אותה בכל מדרגות הטור האחר ותעמיד מה שיצא לך גם כן מסודר בטור רביעי | ||
| + | |- | ||
| + | | | ||
| + | :*The same is done with the third and fourth terms or whichever of the given terms, arranging all as is seen in this diagram: | ||
| + | |style="text-align:right;"|וכן תעשה במדרגה השלישית והרביעית או מה שהנחת מהמדרגות ותסדר זה כלו כמו שאתה מראה בזאת הצורה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color: blue; text-align:center;" | ||
| + | |- | ||
| + | |128||64||32||16||8||4|| || | ||
| + | |- | ||
| + | |384||192||96||48||24||12||3||6 | ||
| + | |- | ||
| + | |640||320||160||80||40||20||5||10 | ||
| + | |- | ||
| + | |896||448||224||112||56||28||7||14 | ||
| + | |- | ||
| + | |1152||576||288||144||72||36||9||18 | ||
| + | |- | ||
| + | |1408||704||352||176||88||44||11||22 | ||
| + | |- | ||
| + | |1664||832||416||208||104||52||13||26 | ||
| + | |- | ||
| + | |1920||960||480||240||120||60||15||30 | ||
| + | |} | ||
| − | ===== | + | | |
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | |קכח||סד||לב||יו||ח||ד|| || | ||
| + | |- | ||
| + | |שפד||קצב||צו||מח||כד||יב||ג||ו | ||
| + | |- | ||
| + | |תרמ||שך||קס||פ||מ||כ||ה||י | ||
| + | |- | ||
| + | |תתצו||תמח||רכד||קיב||נו||כח||ז||יד | ||
| + | |- | ||
| + | |אלף קנב||תקעו||רפח||קמד||עב||לו||ט||יח | ||
| + | |- | ||
| + | |אלף תח||תשד||שנב||קעו||פח||מד||יא||כב | ||
| + | |- | ||
| + | |אלף תרסד||תתלב||תיו||רח||קד||נב||יג||כו | ||
| + | |- | ||
| + | |אלף תתקך||תתקס||תף||רמ||קכ||ס||טו||ל | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | :*A lengthwise line was added breadthwise [column 1], next to the line of the natural odds [column 2], in which the even-times-odd numbers were placed by the order of their terms, starting from 6, in order to demonstrate all the properties mentioned of the three species of even numbers, since this diagram includes them together. | ||
| + | |style="text-align:right;"|וכבר הוספנו ברחב לוח הולך באורך ילוה ללוח אשר בו הנפרד הטבעי העמדנו בו זוג הנפרד על סדר מדרגותיו אשר התחלתם ששה כדי להראות בזה כל מה שזכרנו מהסגולות למיני הזוג השלשה אחר שזאת הצורה תקיף עליהם יחד | ||
| + | |- | ||
| + | | | ||
| + | *[even-times-odd numbers:] | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :*[<math>\scriptstyle{\color{red}{6;\;10;\;14}}</math>] When setting 6 as the smaller extreme of the even-times-odd numbers and 14 as the greater extreme, their sum is 20, and the mean, which is 10, when it is doubled is the same. | ||
| + | ::<math>\scriptstyle{\color{blue}{a_1+a_3=6+14=20=2\sdot10=2a_2}}</math> | ||
| + | |style="text-align:right;"|כי אתה כאשר תשים הקצה הקטן מזוג הנפרד ששה והקצה הגדול ממנו י"ד תמצאם מקובצים כ' ותמצא האמצעי אשר הוא י' כאשר תכפלהו כמו זה | ||
| + | |- | ||
| + | | | ||
| + | :*[<math>\scriptstyle{\color{red}{6;\;10;\;14;\;18}}</math>] When setting 18 as the greater extreme, the sum of the two extremes is 24, and the two means, which are 10 and 14, are also 24. | ||
| + | ::<math>\scriptstyle{\color{blue}{a_1+a_4=6+18=24=10+14=a_2+a_3}}</math> | ||
| + | |style="text-align:right;"|וכן אם שמת הקצה הגדול י"ח תמצא שני הקצוות מקובצים כ"ד וכן שני האמצעיים והם י' וי"ד כ"ד | ||
| + | |- | ||
| + | | | ||
| + | *even-times-even numbers | ||
| + | |style="text-align:right;"|ואמנם זוג הזוג | ||
| + | |- | ||
| + | | | ||
| + | :*[<math>\scriptstyle{\color{red}{4;\;8;\;16}}</math>] When setting 4 as the smaller extreme of [the even-times-even numbers] and 16 as the greater extreme, the product of 4 by 16 is equal to the product of the mean, which is 8, by itself, which is 64. | ||
| + | ::<math>\scriptstyle{\color{blue}{a_1\sdot a_3=4\sdot16=64=8^2=a_2^2}}</math> | ||
| + | |style="text-align:right;"|הנה אתה אם שמת הקצה הקטן ממנו ד' והגדול י"ו תמצא מה שיתקבץ מהכאת ד' בי"ו שוה למה שיתקבץ ממוכה האמצעי והוא ח' בעצמו והוא ס"ד | ||
| + | |- | ||
| + | | | ||
| + | :*[<math>\scriptstyle{\color{red}{4;\;8;\;16;\;32}}</math>] When setting 32 as the greater extreme, the product of 4 by 32 is 128 and it is equal to the product of the two means one by the other, which are 8 and 16. | ||
| + | ::<math>\scriptstyle{\color{blue}{a_1\sdot a_4=4\sdot32=128=8\sdot16=a_2\sdot a_3}}</math> | ||
| + | |style="text-align:right;"|ואם שמת הקצה הגדול ל"ב תמצא כלל הכאת ד' בל"ב קכ"ח והוא שוה למה שיהיה מהכאת שני האמצעיים אחד מהם באחד והם ח' וי"ו | ||
| + | |- | ||
| + | |It has been clarified from the properties of even-times-even-times-odd numbers that were explained before and from what is perceived from this diagram after the completion that: | ||
| + | |style="text-align:right;"|הנה כבר התבאר ממה שבארנו לפנים מסגולות זוג הזוג וזוג הנפרד וממה שנמצאהו משיג לזאת הצורה אחרי השלמה | ||
| + | |- | ||
| + | |<span style=color:red>[odd × even-times-even-times-odd]</span> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | *When multiplying first a term of the natural odd numbers by the first term of the even-times-even-times-odd numbers of whichever of the lengthwise lines starting breadthwise [column], the product is far from that [even-times-even-times-odd] term by two intermediary terms and it is the fourth term starting from that term by which the even-times-even-times-odd terms are multiplied lengthwise. | ||
| + | |style="text-align:right;"|שאתה כשתכה תחלת מדרגה ממדרגות הנפרד הטבעי במדרגה הראשונה ממדרגות זוג הזוג והנפרד מאי זה מן הטורים ההולכים באורך המתחילים מן הרחב שתרצה תמצא ההגעה כבר תתרחק מאותה המדרגה שני אמצעיים והיה הוא המדרגה הרביעית מאותה המדרגה המוכה בה ממדרגות זוג הזוג והנפרד באורך | ||
| + | |- | ||
| + | | | ||
| + | *When multiplying the second term of the odd numbers by the same first term of the even-times-even-times-odd numbers, the product is far from the same first even-times-even-times-odd term [of that column] by five intermediary terms, it is far from the first product [of the first even-times-even-times-odd number of that column by the first odd number] by two intermediary terms, and it is the fourth term starting from the first product [of the first even-times-even-times-odd number of that column by the first odd number] lengthwise. | ||
| + | |style="text-align:right;"|ואם תכה המדרגה השנית ממדרגות הנפרד באותה המדרגה הראשונה ממדרגות זוג הזוג והנפרד תמצא ההגעה מתרחקת מאותה המדרגה הראשונה ממדרגת זוג הזוג והנפרד חמש אמצעיים והיא מתרחקת מההגעה הראשונה שני אמצעיים ותהיה ההגעה השנית היא המדרגה הרביעית מההגעה הראשונה באורך | ||
| + | |- | ||
| + | | | ||
| + | *For each of odd terms, its product [by an even-times-even-times-odd number] is far from the preceding product of the odd number by the same even-times-even-times-odd number by two intermediary terms lengthwise. | ||
| + | |style="text-align:right;"|וכמו כן ישיג כל מדרגות הנפרד שכל מה שתרד בם מדרגה תעבור הגעת אשר לפניה בשני אמצעיים באורך כאשר היתה המדרגה המוכה בה מדרגות הנפרד ממדרגות זוג הזוג והנפרד לבדו | ||
| + | |- | ||
| + | |<span style=color:red>[even-times-even × even-times-even-times-odd]</span> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | *When multiplying the first term of the even-times-even numbers by the first term of the even-times-even-times-odd numbers of whichever of the breadthwise lines, the product is far from that even-times-even-times-odd number by one intermediary term breadthwise. | ||
| + | |style="text-align:right;"|ואמנם אם תכה המדרגה הראשונה מזוג הזוג במדרגה הראשונה מזוג הזוג והנפרד מאי זה מהטורים ההולכים ברחב שתרצה תעבור אמצעי מהמדרגה המוכה בה מזוגות הזוגות והנפרד והיית בעברך האמצעי לוקח ברחב מכוין למה שהיית עושה בהכאת מדרגות הנפרד הנה נפלת על ההגעה אשר תהיה מהכאת המדרגה הראשונה מזוג הזוג במדרגה הא' מאי זה מטורי זוג הזוג והנפרד שתרצה ההולכים ברחב המתחילים בארך | ||
| + | |- | ||
| + | | | ||
| + | *When multiplying the second term of the even-times-even numbers by the same first term of the even-times-even-times-odd numbers, the product is far from the multiplied even-times-even-times-odd term [of that column] by two intermediary terms, and it is the fourth term [starting from this even-times-even-times-odd number] breadthwise. | ||
| + | |style="text-align:right;"|ואם תכה המדרגה השנית ממדרגות זוג הזוג במה שהכית בו ממדרגות זוג הזוג והנפרד ר"ל המדרגה הראשונה מזוג הזוג והנפרד תמצא ההגעה כבר נתרחקה מן המדרגה המוכה בה מזוג הזוג והנפרד שני אמצעיים והייתה המדרגה הרביעית ממנה ברחב | ||
| + | |- | ||
| + | | | ||
| + | ::When multiplying the second term of the even-times-even numbers, which is 8, by the second term breadthwise of the even-times-even-times-odd numbers [the first term in the second column], which is 24, the product [<math>\scriptstyle{\color{red}{8\times24=192}}</math>] is far from 24 by two intermediary terms [breadthwise], yet it is not far by two intermediary terms from the product [of the first term of the even-times-even numbers by the first term in the second column of the even-times-even-times-odd numbers], but from the multiplied [i.e. by the first term in the second column of the even-times-even-times-odd numbers] itself. | ||
| + | |style="text-align:right;"|וגם כן אם תכה המדרגה השנית מזוג הזוג והיא ח' במדרגה השנית ברחב מזוג הזוג והנפרד והיא כ"ד תמצא ההגעה מתרחקת מכ"ד שני אמצעיים גם כן ולא תתרחק מההגעה הראשונה שני אמצעיים אבל מהמוכה בה | ||
| + | |- | ||
| + | |Therefore, it is clear that when the first term of the even-times-even numbers is multiplied by the terms of any column of the even-times-even-times-odd numbers for each descending term, the product moves away by one term breadthwise from the first product. | ||
| + | |style="text-align:right;"|הנה אם כן כבר התבאר שמוכה המדרגה הראשונה ממדרגות זוג הזוג כשהוכה במדרגות טור מטורי זוג הזוג והנפרד ההולכים ברחב כל מה שירד מדרגה ירד אחרת מההגעה הראשונה לוקח ברחב | ||
| + | |- | ||
| + | |Also, when the first term of the even-times-even-times-odd numbers is multiplied by the even-times-even numbers the products are arranged successively term by term breadthwise with no intermediary term in a line starting from an even-times-even-times-odd term. | ||
| + | |style="text-align:right;"|ושהמדרגה הראשונה ממדרגות טורי זוג הזוג והנפרד כאשר תוכה בכל מדרגות זוג הזוג ויצאו ההגעות ימשכו קצתם לקצת בלא אמצעי בטור אשר התחלתו היא המדרגה מזוג הזוג והנפרד | ||
| + | |- | ||
| + | |This is the end of the discussion on the three categories of the even numbers that are evident in the categorization mentioned before. | ||
| + | |style="text-align:right;"|הנה זה סוף המאמר בחלקי הזוג השלשה הנראים בחלוקה אשר זכרנו לפנים | ||
| + | |- | ||
| + | |The mentioning of the perfect, the superabundant, and the deficient even number is in another place that is more appropriate than where the author of the book mentioned it, and there it will be discussed, by the will of God. | ||
| + | |style="text-align:right;"|אמנם זכירת הזוג השוה והנוסף והחסר הוא בזולת זה המקום יותר ראוי באשר זכרו מניח הספר ושם נדבר עליו ברצון השם יתע‫' | ||
| + | |- | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| − | + | ===== The Review of the Odd Number and The Explanation of its Categories ===== | |
| + | |||
| + | |style="text-align:right;"|<big>בזכירת המספר הנפרד ובאור חלוקותיו</big> | ||
| + | |- | ||
| + | |The odd number, as said before, is that which is divided by any division into two unequal parts greater and smaller, and as its division is approaches as closely as possible to equality, one of them exceeds the other by one | ||
| + | |style="text-align:right;"|המספר הנפרד כמו שאמרנו לפנים הוא אשר איך שחולק חלק בשני חלקים מתחלקים בתוספת וחסרון וכשיקרבו חלוקותיו מהשווי תכלית מה שאיפשר היה ביניהם אחד יעדיף אחד מהם על האחר | ||
| + | |- | ||
| + | |Its nature is divided into two types and a third type accidentally occurs | ||
| + | |style="text-align:right;"|והוא יחלק בטבע לשני חלקים ויקרה לו שיהיה לו חלק שלישי | ||
| + | |- | ||
| + | | | ||
| + | *one of the natural two types is called incomposite prime | ||
| + | |style="text-align:right;"|ואמנם האחד מהשנים אשר בטבע יאמר לו ראשון בלתי מורכב | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *the other is called secondary composite [= composite odd] |
| + | |style="text-align:right;"|ואמנם האחר יאמר לו שני מורכב | ||
|- | |- | ||
| | | | ||
| − | ====== Prime Incomposite Number ====== | + | *the third is derived from the relation of both types - when a second composite odd number is compared to a second composite odd number so that one of them is prime and incomposite in relation to the other [= relatively prime] |
| + | |style="text-align:right;"|ואמנם השלישי הנה יקרה משני אלו החלקים בהצטרף והוא שיהיה נפרד שני מורכב יצורף אל נפרד שני מורכב ויהיה כל אחד מהם אצל חבירו ראשון בלתי מורכב | ||
| + | |- | ||
| + | |The [sum] of the terms of the natural odd number is the same as the product of the number of the terms by itself <math>\scriptstyle\sum_{i=1}^n \left(2i-1\right)=n^2</math>. | ||
| + | |style="text-align:right;"|וכבר ישיג הנפרד הטבעי שכל מספר מדרגותיו כמו הכאת מספרי המדרגות בעצמם | ||
| + | |- | ||
| + | |Examine it in this diagram: | ||
| + | |style="text-align:right;"|ותבחן זה בצורה הזאת | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|א' ג' ה' ז' ט' י"א י"ג ט"ו י"ז י"ט כ"א כ"ג כ"ה | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | ====== The Review of the Prime Incomposite Number ====== | ||
| − | + | |style="text-align:right;"|<big>בזכירת הנפרד הראשון הבלתי מורכב<big> | |
| + | |- | ||
| + | |{{#annot:definition|76,2147|1noC}}The first type of odd numbers is incomposite, that has no divisor but the one, such as: 3; 5; 7; 11; 13; 17. These numbers and others like them have no divisors and no fractional parts but the one which [are a part whose name] is derived from the whole number itself. | ||
| + | |style="text-align:right;"|המספר הנפרד הראשון שהוא בלתי מורכב אשר אין לו חלק ימנהו בלתי האחד כמו ג' ה' ז' י"א י"ג י"ז אלו המספרים ומה שימצא כמותם לא ימצא להם מספר ימנם ואין להם חלק כלל ימנם בלתי האחדים שנגזר להם חלק מכלל המספר{{#annotend:1noC}} | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Such as: <math>\scriptstyle{\color{blue}{\frac{1}{3}}}</math>→ from 3; and <math>\scriptstyle{\color{blue}{\frac{1}{7}}}</math>→ from 7 |
| + | |style="text-align:right;"|כמו השלישי מג' והשביעית מז' אשר הוא האחד | ||
| + | |- | ||
| + | |It should be called prime, because it is measured only by the unit. | ||
| + | |style="text-align:right;"|הנה בראוי אם כן נאמר שהוא ראשון אחר שלא ימנהו בלתי האחדות | ||
| + | |- | ||
| + | |It is incomposite, since it cannot be divided into equal numbers [i.e. parts other than ones], whereas it is produced from the sequence of units. | ||
| + | |style="text-align:right;"|ושהוא בלתי מורכב אחר שאי אפשר שיחלק בחלקי מספרים שוים לפי שהוא אמנם התילד מסדר האחדים והמשכם | ||
| + | |- | ||
| + | |An odd number has a divisor if it is a product of this type of odd number, i.e. a prime incomposite odd number. | ||
| + | |style="text-align:right;"|ואמנם יהיה למספר הנפרד חלק ימנהו כאשר היה נכפל מזה המין מהנפרד ר"ל {{#annot:term|76,2147|Wnyo}}הנפרד הראשון הבלתי מורכב{{#annotend:Wnyo}} | ||
| + | |- | ||
| + | | | ||
| + | *If it is multiplied an even number of times, the product is an even number <math>\scriptstyle2m\sdot\left(2n-1\right)</math>. | ||
| + | |style="text-align:right;"|כי אם נכפל פעמים מספרם זוג יתילד מהם מספר הזוג | ||
| + | |- | ||
| + | | | ||
| + | :An even number necessarily has a divisor, even if its divisors are very few, since two is its divisor and the number of times by which two divides it is also its divisor. | ||
| + | |style="text-align:right;"|והמספר הזוג לו מספר ימנהו בהכרח לפי שאפילו ימעטו חלקיו המונים אותו מאד הנה השנים ימנהו וימנהו גם כן כלל אחדי הפעמים אשר בם ימנוהו השנים | ||
| + | |- | ||
| + | | | ||
| + | :*If the even number of times by which the odd number is multiplied is 2 alone, [the product] produced from every odd is an even-times-odd number <math>\scriptstyle2\sdot\left(2n-1\right)</math>. | ||
| + | |style="text-align:right;"|ואם היו הפעמים הזוג אשר נכפל בם הנפרד שנים לבד יתחדש מכל נפרד מהם זוג הנפרד | ||
| + | |- | ||
| + | | | ||
| + | :*If the odd number is multiplied an even number of times and this even number is larger than two, the product is an even-times-even-times-odd number <math>\scriptstyle 2^nm\sdot\left(2n-1\right)</math>. | ||
| + | |style="text-align:right;"|ואם נכפל הנפרד מספר פעמים והיה מספר הפעמים זוג והיה הזוג יותר משנים הנה המתחדש מהם זוג הזוג והנפרד | ||
| + | |- | ||
| + | | | ||
| + | *If it is multiplied an odd number of times, the product is an odd number <math>\scriptstyle\left(2m-1\right)\sdot\left(2n-1\right)</math>, so that the multiplied odd number divides it by the number of times by which it is multiplied, and [the product] is also divided by the number which multiplies the prime incomposite odd number, that is by the multiplied odd number. | ||
| + | |style="text-align:right;"|ואם נכפל פעמים מספרם נפרד יחודש ממנו נפרד ימנהו אותו המספר הנפרד הנכפל בשעור אחדי הפעמים אשר נכפל בם וימנהו ג"כ כלל אחדי הפעמים אשר נכפל בם אותו {{#annot:term|76,2147|Kz95}}המספר הנפרד הראשון הבלתי מורכב{{#annotend:Kz95}} רצוני לומר בשעור אחדי אותו הנפרד הנכפל | ||
| + | |} | ||
| + | {| | ||
|- | |- | ||
| | | | ||
| − | |||
| − | + | ====== The Review of the Secondary Composite Odd Number ====== | |
| + | |||
| + | |style="text-align:right;"|<big>בזכר {{#annot:term|77,2148|f33t}}המספר הנפרד השני המורכב{{#annotend:f33t}}</big> | ||
| + | |- | ||
| + | |{{#annot:definition|77,2148|Txwl}}The composite odd number has a divisor beside one, and that number is a fractional part of it. | ||
| + | |style="text-align:right;"|המספר הנפרד המורכב הוא אשר לו עם האחד מספר ימנהו וזה המספר הוא חלק לו{{#annotend:Txwl}} | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :It was already said that the prime odd number is produced from the sequence of the units alone. |
| + | |style="text-align:right;"|וכבר אמרנו ש{{#annot:term|76,2147|mL7w}}המספר הנפרד הראשון{{#annotend:mL7w}} אמנם התילד מהמשך האחדים לבד | ||
| + | |- | ||
| + | | | ||
| + | :If the number is a multiplied odd number, it is produced from the product of prime odd numbers that are multiplied an odd number of times. | ||
| + | |style="text-align:right;"|ואמנם אם יהיה המספר נפרד נכפל הנה לא יהיה ואמנם זה הנה אמנם יצמח ויתילד מהכפלת הנפרדים הראשונים כשנכפלו פעמים מספרם נפרד | ||
| + | |- | ||
| + | |Hence, this type of odd number is composed of the prime odd number. | ||
| + | |style="text-align:right;"|הנה זה הנפרד אם כן מורכב מ{{#annot:term|76,2147|V8xR}}הנפרד הראשון{{#annotend:V8xR}} | ||
| + | |- | ||
| + | | | ||
| + | *It is secondary, as it is formed from the prime. | ||
| + | |style="text-align:right;"|הנה הוא אם כן שני לפי שהוא אמנם חודש מהראשון | ||
| + | |- | ||
| + | | | ||
| + | *It is composite, as the prime odd number is its fractional part. | ||
| + | |style="text-align:right;"|והוא מורכב לפי שהנפרד הראשון חלק לו | ||
| + | |- | ||
| + | | | ||
| + | ::Such as: 9; 15; 25; 27 | ||
| + | |style="text-align:right;"|וזה כמו ט' וט"ו וכ"ה וכ"ז | ||
| + | |- | ||
| + | | | ||
| + | ::*9 is composed from the multiplication of 3 three times <math>\scriptstyle{\color{blue}{9=3\times3}}</math>. | ||
| + | |style="text-align:right;"|כי ט' מורכב מהכפלת ג' שלשה פעמים | ||
| + | |- | ||
| + | | | ||
| + | ::*15 is composed from the multiplication of 5 three times, or from the duplication of 3 five times <math>\scriptstyle{\color{blue}{15=5\times3=3\times5}}</math>. | ||
| + | |style="text-align:right;"|וט"ו מורכב מהכפלת ה' שלשה פעמים או מהכפלת ג' חמשה פעמים | ||
| + | |- | ||
| + | | | ||
| + | ::*21 is composed from the multiplication of 7 three times, or from the duplication of 3 seven times <math>\scriptstyle{\color{blue}{21=7\times3=3\times7}}</math>. | ||
| + | |style="text-align:right;"|וכמו כן כ"א מהכפלת ז' שלשה פעמים או מכפל ג' שבעה פעמים | ||
| + | |- | ||
| + | |? | ||
| + | |style="text-align:right;"|הנה אם כן כבר יכלול זה המין מהנפרד | ||
| + | |- | ||
| + | |The first species [prime odd number] has a fractional part that is derived from its name, but this is not a number - it is the unity [p has the part 1/p, but this part is not a number, because it is the unit]. This is the property of the genus of genera, which is the number, i.e. that it is somposed of the units. | ||
| + | |style="text-align:right;"|והמין הראשון שיש לו חלק נגזר השם משמו אינו מספר והוא ה{{#annot:term|369,1686|Erja}}אחדות{{#annotend:Erja}} כי זה סגולת סוג הסוגים אשר הוא המספר ר"ל שהוא מורכב מהאחדים | ||
| + | |- | ||
| + | |The secondary composite [odd number] is distinct for having a fractional part that is not derived from its name. | ||
| + | |style="text-align:right;"|ואמנם מה שנתיחד בו {{#annot:term|77|rJHn}}השני המורכב{{#annotend:rJHn}} הנה הוא שיש לו חלק אינו נגזר משמו ימנהו | ||
| + | |- | ||
| + | | | ||
| + | ::Such as: | ||
| + | ::*9 - for even though it has a part that is derived from its name, it is one, which is a ninth of the nine, it has also a third, which is three. | ||
| + | |style="text-align:right;"|כמו ט' כי הוא ואם היה לו חלק נגזר משמו הוא האחד אשר הוא תשיעית התשעה יש לו שליש גם כן הוא השלשה | ||
| + | |- | ||
| + | | | ||
| + | ::*15 - has a fifth, which is 3; a third, 5; as well as one part of 15, which is the one. | ||
| + | |style="text-align:right;"|וכמו כן ט"ו יש לו חומש והוא ג' ושליש ה' עם החלק מט"ו אשר הוא האחד | ||
| + | |} | ||
| + | {| | ||
|- | |- | ||
| | | | ||
| − | |||
| − | + | ====== The Quality of the Third Species of Odd Number - The Relatively Prime Number ====== | |
| + | |||
| + | |style="text-align:right;"|<big>תאר המין השלישי מהמספר הנפרד והוא ראשון אצל האחר</big> | ||
| + | |- | ||
| + | |It was already said in the categorization of the odd number that this type is species arises accidentally, as it is relatively that the two composite numbers have no common number that counts them [= common divisor], but each of them, regarded in its nature, has a number that counts it, which is a part [= divisor] of it. | ||
| + | |style="text-align:right;"|כבר אמרנו בחלוקת המספר הנפרד שזה המין יתחדש לנפרד במקרה לפי שהוא אמנם יהיה עם ההקשה בין שני המספרים המורכבים אין להם מספר משותף ימנה אותם ולכל אחד מהם כאשר הושב אל טבעו מספר ימנהו הוא חלק לו | ||
| + | |- | ||
| + | | | ||
| + | ::Such as 9 that is composed, as said, from the multiplication of 3 three times, with respect to 25, which is composed from 5 five times, each of these two numbers is prime and incomposite relative to its companion, as they have no common number that counts them [=common divisor]. | ||
| + | |style="text-align:right;"|וזה כמו ט' אשר הם מורכבים כמו שאמרנו מהכפלת ג' שלשה פעמים הנה כאשר הוקשו אל כ"ה אשר הם מורכבים מה' חמשה פעמים הנה כל אחד משני אלו המספרים אצל חבירו ראשון בלתי מורכב לפי שאין להם מספר משותף ימנם | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::They would have had such a common number, if the fractional part of one of them were a fractional part of the other, since the fractional part is the counting of the whole. |
| + | |style="text-align:right;"|ואמנם היה שיהיה להם מספר משותף אלו היה החלק לאחד מהם חלק לאחר אחר שהחלק הוא המונה לכל | ||
|- | |- | ||
| | | | ||
| + | ::But the three, which is a fractional part of 9, is not a fractional part of 25 that counts it, nor the five, which is a fractional part of 25, a fractional part of 9 that counts it. | ||
| + | |style="text-align:right;"|ואין השלשה אשר הם חלק לתשעה חלק לחמשה ועשרים שימנום ולא החמשה אשר הם חלק לחמשה ועשרים חלק לתשעה שימנום | ||
| + | |- | ||
| + | | | ||
| + | ::They share the one, which is not a number, but the cause of the number. | ||
| + | |style="text-align:right;"|ואמנם ישתתפו באחד אשר אינו מספר ואמנם הוא עלה למספר | ||
| + | |- | ||
| + | |How are these species, which are the species of the odd number, recognized, and how is each species sorted from the natural odd numbers, so that the prime incomposite is distinguished from the secondary composite? This is done by the technique that '''Eratosthenes''' called the "sieve": | ||
| + | |style="text-align:right;"|אמנם איך יוכרו אלו המינים אשר הם מיני הנפרד ואיך יוברר מין מין מהם מהנפרד הטבעי עד שיודע הראשון הבלתי מורכב מהשני המורכב הנה זה בתחבולה יקראה ארסטשאש ה{{#annot:term|2365,1492|xTKM}}מכבר{{#annotend:xTKM}} | ||
| + | |- | ||
| + | | | ||
| + | :By taking the natural odds, beginning from 3, in their natural succession, as in the table above. | ||
| + | |style="text-align:right;"|והיא שתקח הנפרדים הטבעיים אשר התחלתם ג' על סדרם הטבעי ימשכו קצתם לקצת כפי מה שבלוח אשר למעלה | ||
| + | |- | ||
| + | | | ||
| + | :When one wishes the secondary composite among these odds: | ||
| + | |style="text-align:right;"|וכשתרצה השני המורכב מאלו הנפרדים | ||
| + | |- | ||
| + | | | ||
| + | ::*Starting from the first term and skipping two intermediary terms, so that the fourth term is a secondary composite - the first term is 3, the fourth is 9, which is a secondary composite, as it is composed of 3 three times. | ||
| + | |style="text-align:right;"|תתחיל ותעזוב שני אמצעיים אחר המדרגה הראשונה והמדרגה הרביעית מספר שיני מורכב והמדרגה הראשונה ג' והרביעית ט' והיא שני מורכב לפי שהיא מורכבת מג' שלשה פעמים | ||
| + | |- | ||
| + | | | ||
| + | ::*Skipping again two intermediary terms, so that the fourth term from it is also a secondary composite, which is 15 that is composed of 5 three times, or from 3 five times. | ||
| + | |style="text-align:right;"|עוד עזוב ממנו שני אמצעיים והמדרגה הרביעית גם כן ממנה נפרד מורכב והיא ט"ו מורכב מה' שלשה פעמים או מג' חמשה פעמים | ||
| + | |- | ||
| + | | | ||
| + | ::Proceeding always like this, skipping two intermediary terms and the fourth is a secondary composite odd number, until reaching the end of the given natural odd numbers. | ||
| + | |style="text-align:right;"|וכן תעשה תמיד ותעזוב שני אמצעיים והרביעי מספר נפרד שני מורכב עד שתגיע אל סוף מה שהנחת מהנפרדים הטבעיים | ||
| + | |- | ||
| + | | | ||
| + | ::*Then, starting from five, which is the second term of the natural odds, skipping four intermediary terms after it, so that the sixth term from it is a secondary composite, which is 15. | ||
| + | |style="text-align:right;"|אחר תשוב תתחיל מחמשה והיא המדרגה השנית מהנפרדים הטבעיים ותעזוב אחריה ארבע אמצעיים והמדרגה הששית ממנה נפרד שני מורכב והיא ט"ו | ||
| + | |- | ||
| + | | | ||
| + | ::*Also the sixth term from 15, which is 25 - 25 is composed of 5 five times. | ||
| + | |style="text-align:right;"|עוד המדרגה הששית מט"ו והיא כ"ה וכ"ה מורכב מהכפלת ה' חמשה פעמים | ||
| + | |- | ||
| + | | | ||
| + | ::*Again, skipping four intermediary terms after 25 as well, and the sixth term from it is a secondary composite odd number. | ||
| + | |style="text-align:right;"|עוד תעזוב ארבע אמצעיים גם כן אחר כ"ה והמדרגה הששית ממנה היא מספר נפרד שני מורכב | ||
| + | |- | ||
| + | | | ||
| + | ::Until the given natural odd numbers end. | ||
| + | |style="text-align:right;"|עד שיכלה לאשר הנחת מהמספרים הנפרדים | ||
| + | |- | ||
| + | | | ||
| + | ::Proceeding like this - as long as there is a term after the first, continue skipping two intermediary terms for each term. | ||
| + | |style="text-align:right;"|וכן תעשה כל מה שתרד מן ההתחלה מדרגה תוסיף בהשמטת שני האמצעיים שנים לכל מדרגה | ||
| + | |- | ||
| + | |When one does that and writes the terms of the secondary composite odds that are recognized by this technique, nothing is missing, and what remains of those that are not marked [as composite numbers] after completing this and extracting them according to the described description are the prime incomposite odd numbers. | ||
| + | |style="text-align:right;"|כי כשתעשה זה ורשמת על מדרגת {{#annot:term|77,2148|sCb3}}הנפרד השני המורכב{{#annotend:sCb3}} אשר תעמד עליהם בזאת התחבולה לא יבצר ממך דבר והיה מה שישאר אחר כלותך מזה ולקיחתו כפי זה הרושם אשר תארתי לך מאשר לא יפל בם סימן הם נפרדים ראשונים בלתי מורכבים | ||
| + | |- | ||
| + | | | ||
| + | :Know that when you start from the first term, skipping two intermediary terms between each secondary composite and secondary composite: | ||
| + | |style="text-align:right;"|ודע שכאשר החלות במדרגה הראשונה ותעזוב שני אמצעים בין כל שני מורכב ושני מורכב | ||
| + | |- | ||
| + | | | ||
| + | ::The first composite term is composed of the term, from which it began, multiplied by itself, i.e. by the measure of its units. | ||
| + | |style="text-align:right;"|תהיה המדרגה המורכבת הראשונה תצא מורכבת מן המדרגה אשר ממנה התחילה נכפלת בעצמה ר"ל בשיעור אחדיה | ||
| + | |- | ||
| + | | | ||
| + | ::The second composite term is composed of the first term multiplied by the second term. | ||
| + | |style="text-align:right;"|והמדרגה השנית המורכבת מורכבת מהמדרגה הראשונה נכפלת במדרגה השנית | ||
| + | |- | ||
| + | | | ||
| + | ::The third composite term is composed of the first term multiplied by the third term. | ||
| + | |style="text-align:right;"|והמדרגה השלישית המורכבת היא מורכבת מהמדרגה הראשונה נכפלת במדרגה השלישית | ||
| + | |- | ||
| + | | | ||
| + | :And when you start from the second term, skipping four intermediary terms between each composite and composite, the resulting composite numbers will be composed of the second term, from which you started, multiplied by each of the others. | ||
| + | |style="text-align:right;"|ואם תתחיל מן המדרגה השנית ותדלג ארבע אמצעיים בין כל מורכב ומורכב יהיו המורכבים היוצאים מורכבים מהמדרגה השנית אשר החלות ממנה נכפלת בכל אחד מהאחרים | ||
| + | |- | ||
| + | | | ||
| + | :*Each first composite term that is extracted, is the term, from which [the procedure] starts, multiplied by the first term. | ||
| + | |style="text-align:right;"|וכל מדרגה ראשונה מורכבת שתצא היא מן המדרגה אשר ממנה הוחל נכפלת במדרגה הראשונה | ||
| + | |- | ||
| + | | | ||
| + | :*The second composite term is composed of the second term multiplied by itself. | ||
| + | |style="text-align:right;"|והמורכב השני מורכב מהמדרגה השנית נכפלת בעצמה | ||
| + | |- | ||
| + | | | ||
| + | :*The third composite term is composed of the second term, from which [the procedure] starts, multiplied by the third term from the beginning of the terms, which is the third term, from which [the procedure] starts. | ||
| + | |style="text-align:right;"|והמורכב השלישי מורכב מהמדרגה השנית אשר ממנה הוחל נכפלת במדרגה השלישית מראש המדרגות והיא המדרגה השלישית מאשר ממנה הוחל | ||
| + | |- | ||
| + | |The "sieve" is illustrated in order to examine through it all these properties: | ||
| + | |style="text-align:right;"|וכבר ציירתי לך המכבר לבחון בו כל אלו הענינים | ||
| + | |} | ||
| + | |||
| + | {|class="wikitable" style="margin-left: auto; margin-right: auto; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto; text-align:center;" | ||
| + | |- | ||
| + | |כג | ||
| + | |- | ||
| + | | | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto; text-align:center;" | ||
| + | |- | ||
| + | |colspan="2"|כא | ||
| + | |- | ||
| + | |ז||ג | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto; text-align:center;" | ||
| + | |- | ||
| + | |יט | ||
| + | |- | ||
| + | | | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto; text-align:center;" | ||
| + | |- | ||
| + | |יז | ||
| + | |- | ||
| + | | | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto; text-align:center;" | ||
| + | |- | ||
| + | |colspan="2"|טו | ||
| + | |- | ||
| + | |ה||ג | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto; text-align:center;" | ||
| + | |- | ||
| + | |יג | ||
| + | |- | ||
| + | | | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto; text-align:center;" | ||
| + | |- | ||
| + | |יא | ||
| + | |- | ||
| + | | | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto; text-align:center;" | ||
| + | |- | ||
| + | |colspan="2"|ט | ||
| + | |- | ||
| + | |ג||ג | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto; text-align:center;" | ||
| + | |- | ||
| + | |ז | ||
| + | |- | ||
| + | | | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto; text-align:center;" | ||
| + | |- | ||
| + | |ה | ||
| + | |- | ||
| + | | | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto; text-align:center;" | ||
| + | |- | ||
| + | |ג | ||
| + | |- | ||
| + | | | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto; text-align:center;" | ||
| + | |- | ||
| + | |colspan="2"|מה | ||
| + | |- | ||
| + | |טו||ג | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto; text-align:center;" | ||
| + | |- | ||
| + | |מג | ||
| + | |- | ||
| + | | | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto; text-align:center;" | ||
| + | |- | ||
| + | |מא | ||
| + | |- | ||
| + | | | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto; text-align:center;" | ||
| + | |- | ||
| + | |colspan="2"|לט | ||
| + | |- | ||
| + | |יג||ג | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto; text-align:center;" | ||
| + | |- | ||
| + | |לז | ||
| + | |- | ||
| + | | | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto; text-align:center;" | ||
| + | |- | ||
| + | |colspan="2"|לה | ||
| + | |- | ||
| + | |ז||ה | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto; text-align:center;" | ||
| + | |- | ||
| + | |colspan="2"|לג | ||
| + | |- | ||
| + | |יא||ג | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto; text-align:center;" | ||
| + | |- | ||
| + | |לא | ||
| + | |- | ||
| + | | | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto; text-align:center;" | ||
| + | |- | ||
| + | |כט | ||
| + | |- | ||
| + | | | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto; text-align:center;" | ||
| + | |- | ||
| + | |colspan="2"|כז | ||
| + | |- | ||
| + | |ט||ג | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto; text-align:center;" | ||
| + | |- | ||
| + | |colspan="2"|כה | ||
| + | |- | ||
| + | |ה||ה | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |||
| + | {|class="wikitable" style="color:blue; margin-left: auto; margin-right: auto; text-align:center;" | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto; text-align:center;" | ||
| + | |- | ||
| + | |23 | ||
| + | |- | ||
| + | | | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto; text-align:center;" | ||
| + | |- | ||
| + | |colspan="2"|21 | ||
| + | |- | ||
| + | |7||3 | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto; text-align:center;" | ||
| + | |- | ||
| + | |19 | ||
| + | |- | ||
| + | | | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto; text-align:center;" | ||
| + | |- | ||
| + | |17 | ||
| + | |- | ||
| + | | | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto; text-align:center;" | ||
| + | |- | ||
| + | |colspan="2"|15 | ||
| + | |- | ||
| + | |5||3 | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto; text-align:center;" | ||
| + | |- | ||
| + | |13 | ||
| + | |- | ||
| + | | | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto; text-align:center;" | ||
| + | |- | ||
| + | |11 | ||
| + | |- | ||
| + | | | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto; text-align:center;" | ||
| + | |- | ||
| + | |colspan="2"|9 | ||
| + | |- | ||
| + | |3||3 | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto; text-align:center;" | ||
| + | |- | ||
| + | |7 | ||
| + | |- | ||
| + | | | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto; text-align:center;" | ||
| + | |- | ||
| + | |5 | ||
| + | |- | ||
| + | | | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto; text-align:center;" | ||
| + | |- | ||
| + | |3 | ||
| + | |- | ||
| + | | | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto; text-align:center;" | ||
| + | |- | ||
| + | |colspan="2"|45 | ||
| + | |- | ||
| + | |15||3 | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto; text-align:center;" | ||
| + | |- | ||
| + | |43 | ||
| + | |- | ||
| + | | | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto; text-align:center;" | ||
| + | |- | ||
| + | |41 | ||
| + | |- | ||
| + | | | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto; text-align:center;" | ||
| + | |- | ||
| + | |colspan="2"|39 | ||
| + | |- | ||
| + | |13||3 | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto; text-align:center;" | ||
| + | |- | ||
| + | |37 | ||
| + | |- | ||
| + | | | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto; text-align:center;" | ||
| + | |- | ||
| + | |colspan="2"|35 | ||
| + | |- | ||
| + | |7||5 | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto; text-align:center;" | ||
| + | |- | ||
| + | |colspan="2"|33 | ||
| + | |- | ||
| + | |11||3 | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto; text-align:center;" | ||
| + | |- | ||
| + | |31 | ||
| + | |- | ||
| + | | | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto; text-align:center;" | ||
| + | |- | ||
| + | |29 | ||
| + | |- | ||
| + | | | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto; text-align:center;" | ||
| + | |- | ||
| + | |colspan="2"|27 | ||
| + | |- | ||
| + | |9||3 | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto; text-align:center;" | ||
| + | |- | ||
| + | |colspan="2"|25 | ||
| + | |- | ||
| + | |5||5 | ||
| + | |} | ||
| + | |- | ||
| + | |} | ||
| + | |||
| + | {| | ||
| + | |- | ||
| + | |Since it is already clear how to distinguish the secondary composite numbers from the prime incomposite odds by this "sieve", so that the two natural species among the species of the odd number are recognized, each of them by itself, we shall continue to the recognition of the third accidental species and discover how to distinguish, by relating two numbers, each of which is itself a secondary composite, whether they are prime each with respect to its companion, i.e. whether they have no common number that counts them [=common divisor], or, if they do have a common number that counts them, how it is found. | ||
| + | |style="text-align:right;"|ואחר שכבר התבאר לך איך תברור בזה ה{{#annot:term|2365,1492|TOZ3}}מכבר{{#annotend:TOZ3}} המספרים השניים המורכבים מהנפרדים הראשונים הבלתי מורכבים עד שיוכרו שני המינים הטבעיים ממיני הנפרד כל אחד מהם לבדו נמשיך לזה הכרת המין השלישי המקרי ונגלה איך יודע כשנקיש בין שני מספרים כל אחד מהם בעצמו שני מורכב אם הם עם ההקשה כל אחד מהם אצל חברו ראשון ר"ל שאין להם מספר משותף ימנם ואם היה להם מספר משותף ימנם איך נמצאהו | ||
| + | |- | ||
| + | | | ||
| + | :For, when we wish to know it with regard to two numbers, we subtract from the greater of the given numbers the multiples of the smaller that are contained in it. | ||
| + | |style="text-align:right;"|כי אנו כשנרצה לידע זה בשני מספרים הנה אנו נשליך מגדול המספרים המונחים מה שבו מדמיוני הקטן | ||
| + | |- | ||
| + | | | ||
| + | :And when what remains from the greater is less than the smaller, we subtract from the smaller the multiples of the remainder of the greater. | ||
| + | |style="text-align:right;"|וכאשר ישאר מהגדול פחות מן הקטן נשליך מן הקטן מה שבו מדמיוני הנשאר מהגדול | ||
| + | |- | ||
| + | | | ||
| + | :Then, we subtract from the remainder of the greater the multiples of the remainder of the smaller. | ||
| + | |style="text-align:right;"|עוד נשליך מהנשאר מן הגדול מה שבו מדמיוני הנשאר מהקטן | ||
| + | |- | ||
| + | | | ||
| + | :We do not stop subtracting from the greater the multiples of the smaller, until we reach either to the unity or two numbers such that the smaller of them counts the greater. | ||
| + | |style="text-align:right;"|עוד כן לא נסור מחסר מה שביתר מדמיוני הפחות עד שנגיע אם אל האחדות או אל שני מספרים הקטן מהם ימנה הגדול | ||
| + | |- | ||
| + | | | ||
| + | *If they reach the unity, each of them is a prime incomposite with respect to the other. | ||
| + | |style="text-align:right;"|ואם יגיעו אל האחדות הנה כל אחד מהם אצל האחר ראשון בלתי מורכב | ||
| + | |- | ||
| + | | | ||
| + | *If we reach two numbers such that the smaller of them counts the greater, then the smaller is the common number that counts the two numbers that are related to each other. | ||
| + | |style="text-align:right;"|ואם הגענו אל שני מספרים הקטן מהם ימנה הרב הנה הקטן הוא המספר המשותף אשר ימנה שני המספרים אשר הוקש אחד מהם אל האחר | ||
| + | |- | ||
| + | | | ||
| + | ::Example for numbers that each of them is a secondary composite by itself, but with respect to the other it is prime incomposite: 9 and 25. | ||
| + | |style="text-align:right;"|משל זה במספרים אשר כל אחד מהם בעצמו שני מורכב ובהקש אל האחר ראשון בלתי מורכב מספר ט' וכ"ה | ||
| + | |- | ||
| + | | | ||
| + | ::*Subtracting the nines [= modulo] from 25, 7 remains. | ||
| + | |style="text-align:right;"|שאנו נשליך בכ"ה מדמיוני ט' וישאר ז‫' | ||
| + | |- | ||
| + | | | ||
| + | ::*Subtracting the sevents from 9, 2 remains. | ||
| + | |style="text-align:right;"|נשליך מה שבט' מדמיוני ז' ישאר ב‫' | ||
| + | |- | ||
| + | | | ||
| + | ::*Subtracting the twos from 7, 1 remains. | ||
| + | |style="text-align:right;"|נשליך מה שבז' מדמיוני ב' ישאר אחד | ||
| + | |- | ||
| + | | | ||
| + | ::One counts both, but one is not a number. | ||
| + | |style="text-align:right;"|והאחד ימנה השנים ואין האחד מספר | ||
| + | |- | ||
| + | | | ||
| + | ::Hence, 9 and 25, each is prime incomposite with respect to its companion. | ||
| + | |style="text-align:right;"|הנה ט' וכ"ה כל אחד מהם אצל חברו ראשון בלתי מורכב | ||
| + | |- | ||
| + | | | ||
| + | ::Example for numbers that each of them is a secondary composite by itself, and with respect to each other they have a common number [= common divisor] that counts them, so they are secondary composite by it: 21 and 35. | ||
| + | |style="text-align:right;"|ואמנם דמיון המספרים אשר כל אחד מהם שני מורכב ובהקשה ביניהם להם מספר משותף ימנם והם בו שניים מורכבים הם כמו מספר כ"א ול"ה | ||
| + | |- | ||
| + | | | ||
| + | ::*Subtracting the 21 [= modulo] from 35, 14 remains. | ||
| + | |style="text-align:right;"|כי אנו נשליך מה שבל"ה מדמיוני כ"א וישאר י"ד | ||
| + | |- | ||
| + | | | ||
| + | ::*Subtracting the 14 [= modulo] from 21, 7 remains. | ||
| + | |style="text-align:right;"|עוד נשליך מה שבכ"א מדמיוני י"ד וישאר ז‫' | ||
| + | |- | ||
| + | | | ||
| + | ::7 counts 14, therefore 21 and 35 have a common number that counts them [common divisor], which is 7, and they are relatively secondary composite by it. | ||
| + | |style="text-align:right;"|וז' ימנה י"ד הנה כ"א ול"ה להם מספר משותף ימנה אותם והוא ז' והם בו עם ההקשה שניים מורכבים | ||
| + | |- | ||
| + | |Therefore, it is already clear how the accidental third type is extracted from the categories of the odd number. | ||
| + | |style="text-align:right;"|אם כן כבר התבאר איך יוצא המין השלישי המקרי מחלקי הנפרד | ||
| + | |- | ||
| + | |Thus, the discussion on all the types of the number is complete. | ||
| + | |style="text-align:right;"|והנה נשלם המאמר על מיני המספר יחד | ||
| + | |- | ||
| + | |This is the diagram of the categorization of all that was discussed from the beginning of the book to this point: | ||
| + | |style="text-align:right;"|וזה צורת חלוקת מה שדברנו עליו מתחלת ספרנו עד זה המקום | ||
| + | |- | ||
| + | | | ||
| + | {|class="wikitable" | ||
| + | |- | ||
| + | |style="color:blue;"|64 | ||
| + | |The even-times-even number is the divisible into two halves and each half into halves, until the one, its example: | ||
| + | |even-times-even | ||
| + | |- | ||
| + | |style="color:blue;"|14 | ||
| + | |The even-times-odd number is divisible into two halves once, resulting in an odd number, its example: | ||
| + | |even-times-odd | ||
| + | |- | ||
| + | |style="color:blue;"|24 | ||
| + | |The even-times-even-times-odd number is the divisible [by 2] more than once, which does not end in one but in an odd number, its example: | ||
| + | |even-times-even-times-odd | ||
| + | |- | ||
| + | |style="color:blue;"|11 | ||
| + | |The prime incomposite odd number is that which is not counted by any number at all and has no part but the one and itself, its example: | ||
| + | |prime odd | ||
| + | |- | ||
| + | |style="color:blue;"|15 | ||
| + | |The secondary composite number is that which has a number that counts it, which is its part, and the numbers that count it are only odd numbers, its example: | ||
| + | |secondary composite odd | ||
| + | |- | ||
| + | |style="color:blue;"|9, 25 | ||
| + | |The accidental [?] arises from relating two composite odd numbers that have no relatively common number at all, its example: | ||
| + | | | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | |style="text-align:right;"|ס"ד | ||
| + | |style="text-align:right;"|זוג הזוג הוא המתחלק בשני חצאים וכל חצי לחצאים עד האחד ודימיונו | ||
| + | |style="text-align:right;"|זוג הזוג | ||
| + | |- | ||
| + | |style="text-align:right;"|י"ד | ||
| + | |style="text-align:right;"|הזוג הנפרד הוא הנחלק פעם אחת לשני חצאים ויעמד בנפרד ודמיונו | ||
| + | |style="text-align:right;"|הזוג הנפרד | ||
| + | |- | ||
| + | |style="text-align:right;"|כ"ד | ||
| + | |style="text-align:right;"|זוג הזוג והנפרד הוא הנחלק יותר מפעם אחת ולא יכלה לאחד אלא בנפרד ודמיונו | ||
| + | |style="text-align:right;"|זוג הזוג והנפרד | ||
| + | |- | ||
| + | |style="text-align:right;"|י"א | ||
| + | |style="text-align:right;"|הנפרד הראשון הבלתי מורכב הוא אשר לא ימנהו מספר כלל ואין חלק לו אלא האחת ודמיונו | ||
| + | |style="text-align:right;"|הנפרד הראשון | ||
| + | |- | ||
| + | |style="text-align:right;"|ט"ו | ||
| + | |style="text-align:right;"|הנפרד השני המורכב הוא אשר לו מספר ימנהו יהיה לו חלק ולא יהיה המספר המונה לו אלא נפרד ודמיונו | ||
| + | |style="text-align:right;"|נפרד שני מורכב | ||
| + | |- | ||
| + | |style="text-align:right;"|ט' כ"ה | ||
| + | |style="text-align:right;"|המניין המקרי הוא מהקשת שני מספרי' נפרדים מורכבים אין להם מספר משותף בהצטרף כלל ודמיונו | ||
| + | | | ||
| + | |} | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
===== Second Categorization of Even Numbers ===== | ===== Second Categorization of Even Numbers ===== | ||
| − | + | |style="text-align:right;"|<big>חלוקה שנית למספר הזוג</big> | |
| + | |- | ||
| + | |The even number is divided into three categories: | ||
| + | |style="text-align:right;"|המספר הזוג יחלק לשלשה חלקים | ||
| + | |- | ||
| + | | | ||
| + | *Superabundant number, i.e. the sum of its parts exceeds its whole. | ||
| + | |style="text-align:right;"|אם נוסף ר"ל שהגעת כלל חלקיו מוסיף על כלו | ||
| + | |- | ||
| + | | | ||
| + | *Deficient number, i.e. the sum of its parts is less than its whole. | ||
| + | |style="text-align:right;"|ואם חסר ר"ל שהגעת כלל חלקיו יחסרו מכלו | ||
| + | |- | ||
| + | | | ||
| + | *Perfect number i.e. the sum of its parts is equal to its whole. | ||
| + | |style="text-align:right;"|ואם שוה ר"ל שהגעת כלל חלקיו שוה לכלו | ||
| + | |- | ||
| + | |This third type is as a mean between the first two types, which are as separate extremes, since they are in the greatest degree of distinction in surpassing equality by addition and subtraction. | ||
| + | |style="text-align:right;"|וזה המין השלישי כאמצעי בין שני המינים הראשונים אשר הם בדמות קצוות נבדלים אחר שהם בתכלית ההבדל לעברם השווי בתוספת ובחסרון | ||
| + | |- | ||
| + | | | ||
| + | :*The superabundant number is said to be as an animal whose organs are accidentally additional to the natural organs of itself and of all individuals of its form. | ||
| + | |style="text-align:right;"|ונאמ' במספר הנוסף שהוא כמו הבעל חיים שאיבריו הם נוספים במקרה על האיברים הטבעיים לו ולכל אישי צורתו | ||
| + | |- | ||
| + | | | ||
| + | :::Such as 12, 24, and their like: | ||
| + | |style="text-align:right;"|וזה כמו י"ב וכ"ד והדומה להם | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{\begin{align}&\scriptstyle\left(\frac{1}{2}\sdot12\right)+\left(\frac{1}{3}\sdot12\right)+\left(\frac{1}{4}\sdot12\right)+\left(\frac{1}{6}\sdot12\right)+\left(\frac{1}{12}\sdot12\right)\\&\scriptstyle=6+4+3+2+1=16>12\\\end{align}}}</math> | ||
| + | |style="text-align:right;"|כי לי"ב חצי ושליש ורובע ושתות וחלק מי"ב ואלו החלקים מי"ב הם ששה וארבעה וג' ושנים ואחד וכלל זה י"ו והנה י"ו יותר מי"ב והם חלקיו | ||
| + | |- | ||
| + | | | ||
| + | :*The deficient number differs from the superabundant number by attribute, and it is that whose sum of its parts is less than its whole. | ||
| + | |style="text-align:right;"|אמנם המספר החסר הנה הוא המתחלף בתאר למספר הנוסף והוא אשר הגעת חלקיו פחות מכלו | ||
| + | |- | ||
| + | | | ||
| + | ::It is similar to the animal whose natural organs are less than their balance in each of the individuals of its form by nature. | ||
| + | |style="text-align:right;"|וידמה לזה המספר הבעל חיים שאיבריו הטבעיים חסרים משוויים הנמצא בכל אחדי צורתו בטבע | ||
| + | |- | ||
| + | | | ||
| + | :::Such as 8, 14, and their similar: | ||
| + | |style="text-align:right;"|וזה כמו מספר ח' וי"ד והדומה להם | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{\left(\frac{1}{2}\sdot8\right)+\left(\frac{1}{4}\sdot8\right)+\left(\frac{1}{8}\sdot8\right)=4+2+1=7<8}}</math> | ||
| + | |style="text-align:right;"|כי לשמנה מן החלקים חצי ורביעית ושמינית והם ד' ב' א' וכלם ז' וז' פחות מח' אשר ז' כלל חלקיו | ||
| + | |- | ||
| + | |As it is impossible to grasp the end of the departure from equality by addition in nature, as is said in the introduction of the book, so the deficit of that which has magnitude is relatively unbounded, except by a general expression. | ||
| + | |style="text-align:right;"|וכמו שהיציאה מהשווי בתוספת אי אפשר לעמד על תכליתו בטבע כמו שנאמ' בפתיחת הספר כן החסרון בכמות בעל הגודל בלתי מוגבל התכלית אחר שהוא בהצטרף אלא במאמר כולל | ||
| + | |- | ||
| + | |The equality is one mean between two things whose end is impossible to grasp | ||
| + | |style="text-align:right;"|והשווי אחד אמצעי בין שני דברים אי אפשר לעמד על תכליתם | ||
| + | |- | ||
| + | |So is the multitude of the two types, the superabundant and the deficient, that were mentioned in the nature of the even number. | ||
| + | |style="text-align:right;"|כן רבוי שני המינים אשר זכרנום מהנוסף והחסר בטבע המספר הזוג | ||
| + | |- | ||
| + | |Yet, the perfect is rarely found, not as much as the first two species abound. | ||
| + | |style="text-align:right;"|ואמנם השוה אמנם ימצא מעט לא הרבה כמו שיתרבו שני המינים הראשונים | ||
| + | |- | ||
| + | |The perfect even number is obtained by a technique that will be reviewed now. | ||
| + | |style="text-align:right;"|וזה שאנו אמנם נמצא מהמספר הזוג השוה מה שנגיע אליו לבד בתחבולה אשר נזכרה עתה בג"ה | ||
| + | |- | ||
| + | | | ||
| + | ====== The Discussion on the Quality of the Perfect Number and its Derivation ====== | ||
| + | |style="text-align:right;"|<big>הדבור בתאר המספר השוה והוציאו</big> | ||
| + | |- | ||
| + | |It was already said that the perfect number is that whose sum of its parts is equal to its whole. | ||
| + | |style="text-align:right;"|כבר אמרנו שהמספר השוה הוא אשר הגעת כלל חלקיו שוה לכלו | ||
| + | |- | ||
| + | |This number resembles an animal, whose organs are balanced, balanced in its form. | ||
| + | |style="text-align:right;"|וזה המספר ידמה בעל חיים שוה האיברים ממוצע הצורה | ||
| + | |- | ||
| + | | | ||
| + | :::Such as 6, and 28: | ||
| + | |style="text-align:right;"|וזה כמו מספר ו' ומספר כ"ח | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(\frac{1}{2}\sdot6\right)+\left(\frac{1}{3}\sdot6\right)+\left(\frac{1}{6}\sdot6\right)=3+2+1=6}}</math> | ||
| + | |style="text-align:right;"|כי ששה יש לו חצי ושליש ושתות והם ג' ב' א' וכלם ששה הנה אלו הששה שוים לששה אשר הם חלקיו לא יוסיפו ולא יחסרו | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{\begin{align}&\scriptstyle\left(\frac{1}{2}\sdot28\right)+\left(\frac{1}{4}\sdot28\right)+\left(\frac{1}{7}\sdot28\right)+\left(\frac{1}{14}\sdot28\right)+\left(\frac{1}{28}\sdot28\right)\\&\scriptstyle=14+7+4+2+1=28\\\end{align}}}</math> | ||
| + | |style="text-align:right;"|וכמו כן גם כן כ"ח יש לו מהחלקים חצי ורביעית ושביעית וחלק מי"ד וחלק מכ"ח והם י"ד ז' ד' ב' א' ואלו כלם שוים למספר כ"ח אשר הם כלל חלקיו לא יוסיפו ולא יחסרו מהם | ||
| + | |- | ||
| + | |How these perfect numbers are produced, how we recognize their number and how many of them there are in every given [rank] - it is as described: | ||
| + | |style="text-align:right;"|ואמנם איך יתילדו אלו המספרים השווים ואיך נעמוד על המספר הנמצא מהם וכמה מספרים ימצאו מהם בכל מספר מונח הנה הוא במה שאתאר | ||
| + | |- | ||
| + | | | ||
| + | :*Setting the terms of the even-times-even numbers, beginning from one, in a line | ||
| + | |style="text-align:right;"|וזה שנניח מדרגות מספרי זוג הזוג אשר התחלתם מהאחד בטור | ||
| + | |- | ||
| + | | | ||
| + | ::The first term is 1, and the second is 2 - we leave aside the first term and start from 2, setting beneath it the sum that is summed from it and from what precedes it, which is 3. | ||
| + | |style="text-align:right;"|ותהיה המדרגה הראשונה א' והשנית ב' ונעזוב המדרגה הראשונה עוד נתחיל מב' ונניח תחתיה כלל מה שיתקבץ ממנה וממה שלפניה וזה ג‫' | ||
| + | |- | ||
| + | | | ||
| + | ::The same is done for the following terms of the even-times-even - setting beneath each term the sum that is arises from it and from what precedes it. | ||
| + | |style="text-align:right;"|וכן תעשה במה שילוה לה מן המדרגות זוג הזוג תניח תחת כל מדרגה כלל מה שיתקבץ ממנה וממה שלפניה | ||
| + | |- | ||
| + | | | ||
| + | :*Thus, the numbers that are set beneath the terms of the even-times-even are necessarily odd, since the first term is one, and the terms that precede it are even numbers, and the even number, when one is added to it, becomes odd. | ||
| + | |style="text-align:right;"|הנה בהכרח שיהיו המספרים המונחים תחת מדרגות זוג הזוג נפרדים לפי שהמדרגה הראשונה האחד והמדרגות אשר אחריה זוגות והזוג כאשר יוסף עליו האחד ישוב נפרד | ||
| + | |- | ||
| + | | | ||
| + | ::From these odd numbers all the prime incomposite numbers are distinguished, and marked, and the others are left aside. | ||
| + | |style="text-align:right;"|הנה יוכרו מאלו המספרים הנפרדים כל {{#annot:term|76,2147|ZBCw}}מספר ראשון הבלתי מורכב{{#annotend:ZBCw}} ונרשום עליו רושם ונעזוב מה שזולתו | ||
| + | |- | ||
| + | | | ||
| + | :*Then, every marked number is multiplied by what is above it, i.e. the even number, beneath which the prime incomposite odd number is, and the product is set beneath that prime incomposite odd number, so the products are arranged according to their terms respectively in a third line. | ||
| + | |style="text-align:right;"|עוד נכה כל מספר שרשמנו עליו במה שלמעלה ממנו עליו ר"ל במספר הזוג אשר תחתיו אותו הנפרד הראשון הבלתי מורכב ונעמיד מה שיצא מן ההכאה תחת אותו הנפרד הראשון הבלתי מורכב כדי שיסודרו גם כן הגעות המוכים כפי מדרגותיהם בטור שלישי | ||
| + | |- | ||
| + | |When searching by this technique all the terms of the even-times-even numbers that you set, you extract all the perfect numbers that are included in the last term, these are the numbers that are set in the third line, beneath the prime incomposite odds and they are following successively by their natural order, no perfect number among them is skipped. | ||
| + | |style="text-align:right;"|וכאשר תחפש בזאת התחבולה כל מה שהנחת ממדרגות מספרי זוג הזוג הנה כבר הוצאת כל המספרים השלמים אשר הם נכללים תוך מספר המדרגה האחרונה ממה שהוצאת מהם ואותם המספרים הם אשר העמדתם בטור השלישי תחת {{#annot:term|76,2147|rgv9}}הנפרדים הראשונים הבלתי מורכבים{{#annotend:rgv9}} והם נלוים על משך סדרם בטבע לא ידולג ביניהם מספר שלם כלל | ||
| + | |- | ||
| + | |The units that exceed the tens of the perfect numbers are necessarily 6 and 8 alternately - these numbers are always found with this quality. | ||
| + | |style="text-align:right;"|וכבר יתחייב לאלו המספרים השלמים שיהיה הפרט העודף בם על עשרה פעם ו' פעם ח' וכן תמיד ימצאו אלו המספרים בזה התאר | ||
| + | |- | ||
| + | |Examine all that was reviewed concerning their quality and their extraction method in this diagram. | ||
| + | |style="text-align:right;"|ובחן כל מה שזכרתי לך מתארם ודרך הוצאתם בזאת הצורה בג"ה | ||
| + | |- | ||
| + | | | ||
| + | {|class="wikitable" style="color: blue; text-align:center;" | ||
| + | |- | ||
| + | |32||16||8||4||2||1 | ||
| + | |- | ||
| + | |63||31||15||7||3|| | ||
| + | |- | ||
| + | | ||496|| ||28||6|| | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | |לב||יו||ח||ד||ב||א | ||
| + | |- | ||
| + | |סג||לא||טו||ז||ג|| | ||
| + | |- | ||
| + | | ||תצו|| ||כח||ו|| | ||
| + | |} | ||
| + | |||
| + | |} | ||
| + | |||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | === The Discussion of the Quality of the Relative Quantity and its Division into the Equal and the Unequal === | ||
| + | |||
| + | |style="text-align:right;"|<big>הדבור בתאר {{#annot:term|107,2271|k4hQ}}הכמה הצירופיי{{#annotend:k4hQ}} והחלקו אל השוה ובלתי שוה</big> | ||
| + | |- | ||
| + | |After elaborating the discussion on the absolute quantity, completing its discription and the explanation of its interprations, now the relative quantity will be discussed and described properly in accordance with what was described beforehand | ||
| + | |style="text-align:right;"|ואחר אשר הרחבנו המאמר על {{#annot:term|34,1336|GIll}}הכמה הנפרד{{#annotend:GIll}} והשלמנו תאריו ובאור פירושיו אנו נקח עתה לדבר בכמה המצטרף ונתארהו תאר נאות ומסכים למה שתארנו בו מה שקדם לפניו | ||
| + | |- | ||
| + | |The relative quantity is divided in the first division into two parts: one is the equality, and the other is inequality. | ||
| + | |style="text-align:right;"|ו{{#annot:term|107,2579|mNP3}}הכמה המצטרף{{#annotend:mNP3}} יחלק חלוקה ראשונה לשני חלקים אחד מהם השווי והאחר לא שווי | ||
| + | |- | ||
| + | |For, every number is related to a number that is either equal to it, or unequal, with no third species in this division at all. | ||
| + | |style="text-align:right;"|כי כל מספר יחובר אל מספר אם שיהיה שוה לו ואם שיהיה מבלתי שוה מבלתי שיהיה בזאת החלוקה מין שלישי כלל | ||
| + | |- | ||
| + | |There is no supplement nor deficiency in equality, but one of the two relata is equal to the other. | ||
| + | |style="text-align:right;"|הנה השווי אין תוספת בו ולא חסרון אבל יהיה בו אחד מן ב' המצטרפים שוה לאחר | ||
| + | |- | ||
| + | | | ||
| + | :As the hundred, when related to the hundred, the ten to the ten, | ||
| + | |style="text-align:right;"|כמו המאה כאשר הוקשו אל המאה והעשרה אל העשרה | ||
| + | |- | ||
| + | | | ||
| + | :And the similar of the equal to what is related to it among those that are related to it by matter of quantity, whose property is "equal" and "unequal". | ||
| + | |style="text-align:right;"|והדומה לזה ממה שהוא שוה למה שהוקש אליו ממה שהוקשו אליו בענין הכמה אשר סגולתו שוה ולא שוה | ||
| + | |- | ||
| + | |The evenness is not to be divided into species at all, since the matter of evenness is the equal, the equal is equal to the equal, and the even is even to its even. | ||
| + | |style="text-align:right;"|והישר לא יחלק למינים כלל לפי שאמנם ענין הישר השוה והשוה שוה לשוה והישר ישר למיושר לו | ||
| + | |- | ||
| + | |The species of the given numbers that differ in comparison with one another, in inequality, is divided by a second division into two parts - one of them is greater, and the other is smaller, and they are different by name and antithetical by matter. | ||
| + | |style="text-align:right;"|ואמנם המספרים המונחים המתחלפים עם הצטרף קצתם אל קצת ביציאה מהשווי הנה מינם יחלק בחלוקה השנית לשני חלקים אחד מהם גדול והאחר קטן והם מתחלפים בשם ונבדלים בענין | ||
| + | |- | ||
| + | |The greater relation is divided in a third division into five parts: the multiple to the related to it, the superpaticular to the related to it, the superpartient to the related to it, the multiple superparticular to the related to it, and the multiple superpartient to the related to it. | ||
| + | |style="text-align:right;"|וההצטרפות הגדול יחלק בחלוקה השלישית לחמשה חלקים מהם בעל הכפלים למה שהוקש אליו ומהם המוסיף חלק למה שהוקש אליו ומהם המוסיף חלקים למה שהוקש אליו ומהם בעל הכפלים המוסיף חלק למה שהוקש אליו ומהם בעל הכפלים המוסיף חלקים למה שהוקש אליו | ||
| + | |- | ||
| + | |The smaller is divided, like the greater related to it, into five species, each species is parallel to a species of the greater, ascribed and named according to its counterpart, i.e. the species of the smaller are called: the submultiple, the subsuperpaticular, the subsuperpartient, the submultiple-superparticular, and the submultiple-superpartient. | ||
| + | |style="text-align:right;"|ואמנם הקטן יחלק כמו הגדול ההקש בו לחמשה מינים יקביל כל מין מהם מין מהגדול ייוחס ויקרא בהקבלתו ר"ל שיקראו מיני הקטן כשיאמר מין תחת בעל הכפלים ומין תחת המוסיף חלק ומין תחת המוסיף חלקים ומין תחת בעל הכפלים המוסיף חלק ומין תחת בעל הכפלים המוסיף חלקים | ||
| + | |- | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | ==== [Simple Ratios] ==== | ||
| + | |||
| + | | | ||
| + | |- | ||
| + | |Said '''Abū Yusūf''': two of these five ratios are simple: the multiple ratio and the superparticular ratio | ||
| + | |style="text-align:right;"|<big>אמר אבו יוסף</big> ומאלו היחסים החמש שנים מהם פשוטים והם בעל הכפלים והמוסיף חלק | ||
| + | |- | ||
| + | | | ||
| + | :He did not mean by superparticular ratio the multiple superparticular ratio, but he meant the ratio between the two numbers, of which the greater is as the smaller once and as a part of the smaller. | ||
| + | |style="text-align:right;"|ולא ירצה במוסיף חלק בעל הכפלים המוסיף חלק אמנם רצה היחס אשר בין שני המספרים אשר הגדול מהם כמו הקטן פעם אחת וכמו חלק מהקטן | ||
| + | |- | ||
| + | |This is clear in what was clarified for you by '''Plato''', in the book that is attributed to the causes of the capacities that are ascribed to the supreme individuals. | ||
| + | |style="text-align:right;"|וזה מבואר במה שביארו אליך אפלטון בספר המיוחס אל עלות הכחות המיוחסות אל האישים העליונים | ||
| + | |- | ||
| + | | | ||
| + | :This is because he mentioned the numbers that are ascribed to the nine spheres, and related them to each other, relating to each supreme sphere, conceived by the intellect, their natures, of which the numbers ascribed, are of the numbers that are given to the spheres of the four elements. | ||
| + | |style="text-align:right;"|וזה שלמה שזכר המספרים המיוחסים אל הכדורים התשעה וייחס ביניהם לחבר אל כל כדור עליון הדמוי בשכל מטבעיהם מה שיוחסו מספריהם מהמספרים המונחים לכדורי היסודות הארבעה | ||
| + | |- | ||
| + | | | ||
| + | :*to the sphere of the earth and the water - 24 | ||
| + | |style="text-align:right;"|וייחס אל כדור הארץ והמים כ"ד | ||
| + | |- | ||
| + | | | ||
| + | :*to the sphere of the fire and the air - 27 | ||
| + | |style="text-align:right;"|וייחס אל כדור האש והאויר כ"ז | ||
| + | |- | ||
| + | | | ||
| + | :*the sphere of the moon - 36 | ||
| + | |style="text-align:right;"|וייחס אל כדור הירח ל"ו | ||
| + | |- | ||
| + | | | ||
| + | :*the sphere of Mercury and Venus - 48 | ||
| + | |style="text-align:right;"|וייחס אל כדור כוכב ונוגה מ"ח | ||
| + | |- | ||
| + | | | ||
| + | ::Since, as he explained why the sphere of these two planets is one and one number is ascribed to them by saying that these two stars are included in one matter, which is that their distance from the sun is only in the spheres of their motion, and the sum of the movements of their centers and the sun is one, therefore one number is ascribed to them. | ||
| + | |style="text-align:right;"|אחר שבאר למה היו שני אלו הכוכבים כדורם אחד והמספר המיוחס אליהם אחד בשאמר ששני אלו הכוכבים יכללם ענין אחד והוא שמרחקיהם מן השמש אמנם הוא בגלגלי הקפותיהם לבד ואמנם מרכזיהם הנה סך תנועותיהם והשמש אחד ולזה נתייחס אליהם מספר אחד | ||
| + | |- | ||
| + | | | ||
| + | :*the sphere of the sun - 54 | ||
| + | |style="text-align:right;"|ונתייחס אל כדור השמש נ"ד | ||
| + | |- | ||
| + | | | ||
| + | :*the sphere of the Mars - 64 | ||
| + | |style="text-align:right;"|ולכדור מאדים ס"ד | ||
| + | |- | ||
| + | | | ||
| + | :*the sphere of the Jupiter - 72 | ||
| + | |style="text-align:right;"|ולכדור צדק ע"ב | ||
| + | |- | ||
| + | | | ||
| + | :*the sphere of the Saturn - 96 | ||
| + | |style="text-align:right;"|ולכדור שבתאי צ"ו | ||
| + | |- | ||
| + | | | ||
| + | :*the sphere of the fixed stars - 108 | ||
| + | |style="text-align:right;"|ולכדור הכוכבים הקיימים ק"ח | ||
| + | |- | ||
| + | | | ||
| + | :His theory about the attributions to these spheres, that ascribed these numbers, was not שמ accident, without a nesessary reason, but it resulted from a necessary discussion that proves that this is the primary among the description of the relations of these numbers to these spheres in this ratio. | ||
| + | |style="text-align:right;"|ולא היתה הנחתו לאלו היחסים אל אלו הכדורים אשר חייבו זה המספר בהזדמן מבלתי טעם ראוי אבל אמנם נתחייב זה במאמר הכרחי המאמת שזה הוא היותר ראשון במה שיתואר מהצטרפות אלו המספרים אל אלו הכדורים בזה היחס | ||
| + | |- | ||
| + | | | ||
| + | :When he related these numbers, he mentioned that <math>\scriptstyle{\color{blue}{108:24}}</math> is not a simple ratio. | ||
| + | |style="text-align:right;"|וכאשר ייחס בין אלו המספרים זכר שק"ח מכ"ד אינו ביחס פשוט | ||
| + | |- | ||
| + | | | ||
| + | :'''He''' [= Nicomachus] '''said''': because of this we say that the fixed stars are far from the corporeal activities that have essence. | ||
| + | |style="text-align:right;"|<big>אמר</big> ולזה נאמר שהכוכבים הקיימים רחוקים מהפעולות הגשמיות בעלות ההויה | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{108=\left(4\sdot24\right)+\left(\frac{1}{2}\sdot24\right)}}</math> → this is the double sesquialter ratio, which is not a simple ratio. | ||
| + | |style="text-align:right;"|וק"ח כמו ארבעה דמיוני כ"ד וכמו חציים וזהו הכפל המוסיף חלק הנה לא יהיה זה היחס פשוט | ||
| + | |- | ||
| + | |This explains that he did not mean the multiple superparticular ratio by superparticular ratio. | ||
| + | |style="text-align:right;"|זהו ביאור שלא רצה במוסיף חלק בעל הכפלים המוסיף חלק | ||
| + | |- | ||
| + | |I did not elaborate this discussion, thinking that similar discussions by the author were [not] unknown to you, in your casuistry and diligence when studying his discussion and your love to this craft, all the more so as you are among those who have his books | ||
| + | |style="text-align:right;"|ולא הארכתי בזה המאמר הנה לחשבי שכמו זה ממאמרי המחבר נעלם ממך עם פילפולך ושקידתך בעיון דבריו ואהבתך לזאת המלאכה וכל שכן שאתה ממי שספריו אצלו | ||
| + | |- | ||
| + | |But I wanted to remind you of it. | ||
| + | |style="text-align:right;"|אבל ראיתי להזכירך זה | ||
| + | |- | ||
| + | |I have no doubt that our book will fall into the hand of someone who is ignorant of the teacher's opinion, which you yourself know. | ||
| + | |style="text-align:right;"|ולא אספק שספרנו זה יפול אצל מי שסכל מסברת המלמד מה שתדעהו אתה | ||
| + | |- | ||
| + | |And when the discussion has no capacity to yield the essence of the intepretation, the thoughts become confused, the conception collapses, the truths disappear, and knowledge is absent. | ||
| + | |style="text-align:right;"|וכאשר לא היה בכח המאמר שיביא אל האמתת הדרוש יתבלבלו המחשבות ויפול הדמוי ויעלמו האמתיות ויעדרו הידיעות | ||
| + | |- | ||
| + | |May God lead you straight in the light of his explanation, to reach the brightness of his high rank. | ||
| + | |style="text-align:right;"|יישירך האל לאור באורו ולהשיג זיו יקרו | ||
| + | |- | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | ===== The Discussion of the Multiple Ratio - its Nature and its Production ===== | ||
| + | |||
| + | |style="text-align:right;"|<big>הדבור ביחס בעל הכפלים וטבעו והתילדו</big> | ||
| + | |- | ||
| + | |Now, we shall begin with the discussion on the multiple ratio, since it is anterior by nature to the other four remaining ratios. | ||
| + | |style="text-align:right;"|ואמנם עתה נקדים המאמר על {{#annot:term|124,1284|3b4R}}יחס בעל הכפלים{{#annotend:3b4R}} אחר שהוא היותר קודם בטבע משאר היחסים הארבעה הנשארים | ||
| + | |- | ||
| + | | | ||
| + | *When relating the beginning of the numbers, which is 2, to 1, which is the cause of the number, we find that it is double, and this is called the double ratio. <math>\scriptstyle{\color{blue}{2:1}}</math> | ||
| + | |style="text-align:right;"|וזה שאנו כאשר הקשנו תחלת המספרים אשר הוא ב' אל האחד אשר הוא עלת המספר מצאנוה כפל לו וזה הוא הנקרא {{#annot:double ratio|124|EgW9}}הכפל השניי{{#annotend:EgW9}} | ||
| + | |- | ||
| + | | | ||
| + | *If we then relate 1 to the second number, which is 3, the triple ratio arises. <math>\scriptstyle{\color{blue}{3:1}}</math> | ||
| + | |style="text-align:right;"|ואם הקשנו האחד גם כן אל המספר השני אשר הוא ג' יתחדש {{#annot:triple ratio|124|jNnr}}הכפל השלישי{{#annotend:jNnr}} | ||
| + | |- | ||
| + | | | ||
| + | *If we relate it to the third number, which is 4, the quadruple ratio arises. <math>\scriptstyle{\color{blue}{4:1}}</math> | ||
| + | |style="text-align:right;"|ואם הקשנוהו אל המספר השלישי והוא ד' יתחדש {{#annot:quadruple|124|364l}}הכפל הרביעי{{#annotend:364l}} | ||
| + | |- | ||
| + | | | ||
| + | *Likewise when it is related to the terms of the natural numbers, the multiple ratios are created, named by the number to which the one is related. <math>\scriptstyle{\color{blue}{n:1}}</math> | ||
| + | |style="text-align:right;"|עוד כן כאשר יוקש אל מדרגות {{#annot:term|2577,2575|j2Pu}}המספרים הטבעיים{{#annotend:j2Pu}} יתחדשו כפליים יקרא בשם המספר אשר אליו יוקש האחד | ||
| + | |- | ||
| + | |It is clarified that the first ratio that subsists in number is the double ratio, when the beginning of the numbers, which is 2, relates to the one, whose duplication generates the number. | ||
| + | |style="text-align:right;"|הנה התבאר שהיחס הראשון שיפל במספר הוא יחס הכפלים בהצטרף תחלת המספרים אשר הוא ב' אל האחד אשר מהכפלו יהיה המספר | ||
| + | |- | ||
| + | |It is clarified also that the double ratio is the first of the multiple ratios by nature, as it arises by relating the one to the beginning of the numbers. | ||
| + | |style="text-align:right;"|והתבאר שהכפל השניי ראשון למיני הרב הכפליים בטבע לפי שהוא מהקשת האחד אל תחלת המספרים | ||
| + | |- | ||
| + | |The species of the multiple ratios are endless in potentia, as they abound with the abundence of the natural numbers. | ||
| + | |style="text-align:right;"|ואין למיני הכפלים תכלית בכח להתרבותם עם התרבות המספרים הטבעיים | ||
| + | |- | ||
| + | |We will start first with the description of the production of the multiples, setting aside the other of the ratios | ||
| + | |style="text-align:right;"|ונתחיל ראשונה בספור התילד {{#annot:term|124,1284|KPTs}}בעל הכפלים{{#annotend:KPTs}} בלתי שאר היחסים | ||
| + | |- | ||
| + | | | ||
| + | *We begin with the first by nature: | ||
| + | |style="text-align:right;"|ונקדים ממנו הראשון מהם בטבע | ||
| + | |- | ||
| + | | | ||
| + | :The double ratio is that which is generated by relating the double of any number to that number: | ||
| + | |style="text-align:right;"|ונאמר שהכפל השניי הוא אשר יתחדש מיחס כפל כל מספר אליו | ||
| + | |- | ||
| + | | | ||
| + | :Such as: <math>\scriptstyle{\color{blue}{4:2}}</math> and <math>\scriptstyle{\color{blue}{8:4}}</math> | ||
| + | |style="text-align:right;"|כמו ד' אצל ב' וכמו ח' אצל ד‫' | ||
| + | |- | ||
| + | | | ||
| + | :Hence, it is already clarified how the double ratio grows: | ||
| + | |style="text-align:right;"|הנה אם כן כבר התבאר איך יצמח הכפל השניי | ||
| + | |- | ||
| + | | | ||
| + | :*The natural numbers are lined up by the succession of the natural order. | ||
| + | |style="text-align:right;"|וזה כשיסודרו המספרים על משך סדר הטבע | ||
| + | |- | ||
| + | | | ||
| + | ::Such as: 1; 2; 3; 4; 5; 6; 7; as much as one wishes to set. | ||
| + | |style="text-align:right;"|כמו א' ב' ג' ד' ה' ו' ז' עד מה שנרצה להניח | ||
| + | |- | ||
| + | | | ||
| + | :*The natural even numbers are lined up by the succession of the natural order in a line parallel to the first line. | ||
| + | |style="text-align:right;"|עוד נסדר הזוגות הטבעיים על משך סדר הטבע בטור נכחיי לטור הראשון | ||
| + | |- | ||
| + | | | ||
| + | ::Such as: 2; 4; 6; 8; 10; 12; 14 | ||
| + | |style="text-align:right;"|כמו ב' ד' ו' ח' י' י"ב י"ד | ||
| + | |- | ||
| + | | | ||
| + | ::Its terms are as the number of the terms that were set in the line of the sequence of the natural numbers. | ||
| + | |style="text-align:right;"|ויהיו מדרגותיו כמספר המדרגות אשר סדרת אותם בטור {{#annot:term|2576,2575|L8bg}}סדר המספרים הטבעיים{{#annotend:L8bg}} | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color: blue; text-align:center;" | ||
| + | |- | ||
| + | |10||9||8||7||6||5||4||3||2||1 | ||
| + | |- | ||
| + | |20||18||16||14||12||10||8||6||4||2 | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | |י||ט||ח||ז||ו||ה||ד||ג||ב||א | ||
| + | |- | ||
| + | |כ||יח||יו||יד||יב||י||ח||ו||ד||ב | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | :*Then, the first of the terms of the natural evens is related to the first of the terms of the natural numbers; the second to the second; the third to the third; and so on in what follows - we find all of them together in the double ratio. | ||
| + | |style="text-align:right;"|עוד תקיש הראשון ממדרגות הזוג הטבעי בראשון ממדרגות {{#annot:term|2577,2575|pnyj}}המספר הטבעי{{#annotend:pnyj}} והשני בשני והשלישי בשלישי וכן במה שאחר זה שאנו נמצאם יחד ב{{#annot:double ratio|124|m3z8}}יחס הכפל השני{{#annotend:m3z8}} | ||
| + | |- | ||
| + | | | ||
| + | ::Such as: <math>\scriptstyle{\color{blue}{4:2}}</math>; <math>\scriptstyle{\color{blue}{6:3}}</math>; <math>\scriptstyle{\color{blue}{8:4}}</math>; and so on. | ||
| + | |style="text-align:right;"|כמו ד' אצל ב' וו' אצל ג' וח' אצל ד' וכן כלם | ||
| + | |- | ||
| + | | | ||
| + | *The triple ratio is that in which the greater among the two given numbers is thrice the smaller number. | ||
| + | |style="text-align:right;"|אמנם הכפל השלישי הוא שיהיה בגדול משני המספרים המונחים שלשה דמיוני המספר הקטן | ||
| + | |- | ||
| + | | | ||
| + | :Hence, it is clarified how the triple ratio is produced: | ||
| + | |style="text-align:right;"|אם כן הוא מבואר לך איך יתילד הכפל השלישי | ||
| + | |- | ||
| + | | | ||
| + | :*The natural numbers are lined up by the succession of the natural order. | ||
| + | |style="text-align:right;"|וזה כשתסדר המספרים כטבעיים על משך סדר הטבע בטור | ||
| + | |- | ||
| + | | | ||
| + | ::Such as: 1; 2; 3; 4; 5; 6; 7; 8; 9 | ||
| + | |style="text-align:right;"|כמו א' ב' ג' ד' ה' ו' ז' ח' ט‫' | ||
| + | |- | ||
| + | | | ||
| + | :*Then, two terms are skipped and the third is taken and placed at the beginning of another line. | ||
| + | |style="text-align:right;"|עוד נתחיל ונעבור שני מדרגות ונקח השלישית ונשימה ראש מדרגות טור אחד | ||
| + | |- | ||
| + | | | ||
| + | ::The two terms that follow this term are skipped and the third is kept and placed second in the other line. | ||
| + | |style="text-align:right;"|עוד נעבור גם כן שתי מדרגות אחר אותה המדרגה תקח המדרגה השלישית ונשימה ראש מדרגות השנית מהטור האחר | ||
| + | |- | ||
| + | | | ||
| + | ::Likewise, the two terms that follow each taken term are skipped and the third is kept and set in order among the terms of the other line. | ||
| + | |style="text-align:right;"|עוד כן נדלג שתי מדרגות אחר כל מדרגה שנקח ונחזיק בשלישית ממנה ונסדרם במדרגות אשר בטור האחר | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color: blue; text-align:center;" | ||
| + | |- | ||
| + | |10||9||8||7||6||5||4||3||2||1 | ||
| + | |- | ||
| + | |30||27||24||21||18||15||12||9||6||3 | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | |י||ט||ח||ז||ו||ה||ד||ג||ב||א | ||
| + | |- | ||
| + | |ל||כז||כד||כא||יח||טו||יב||ט||ו||ג | ||
| + | |} | ||
| + | |||
| + | |- | ||
| + | | | ||
| + | :*Thereafter, the [first] term of the resulted line is related to the first term of the natural numbers; the second to the second; the third to the third - we find them in the triple ratio. | ||
| + | |style="text-align:right;"|ואחר נקיש בין המדרגות מהטור המוצא ובין המדרגה הראשונה מטור {{#annot:term|2577,2575|8Qcb}}המספר הטבעיי{{#annotend:8Qcb}} עוד השנית בשנית והשלישית בשלישית הנה אנו נמצאם ב{{#annot:triple ratio|124|YI1E}}יחס הכפל השלישי{{#annotend:YI1E}} | ||
| + | |- | ||
| + | | | ||
| + | ::Such as: <math>\scriptstyle{\color{blue}{3:1}}</math>; <math>\scriptstyle{\color{blue}{6:2}}</math>; <math>\scriptstyle{\color{blue}{9:3}}</math>; and so on until the end of the numbers that were set. | ||
| + | |style="text-align:right;"|כמו ג' אצל א' ו' אצל ב' וט' אצל ג' וכן תמיד עד סוף שהנחת מן המספרים | ||
| + | |- | ||
| + | | | ||
| + | :Thus it is clear that the triple ratio is generated by multiplying each of the terms of the natural numbers by three and placing these products as terms of another line. | ||
| + | |style="text-align:right;"|הנה אם כן כבר התבאר שאמנם יתחדש {{#annot:triple ratio|124|35hm}}יחס הכפל השלישיי{{#annotend:35hm}} מהכאת כל מדרגה ממדרגות המספרים הטבעיים בשלשה והעמדת אלו ההגעות במדרגות טור אחר | ||
| + | |- | ||
| + | | | ||
| + | :It is necessary for the line of the greater numbers in this ratio, and any ratio that is derived from multiplication of numbers by an odd number, that it will be [as follows:] an odd number, then an even number, then another odd number, as the observation shows. | ||
| + | |style="text-align:right;"|וכבר יתחייב לטור אשר בו מדרגות המספרים הגדולים מזה היחס ובכל יחס נגזר ההכפלה ממספר נפרד אל מספר שיהיה נפרד ואחר כן זוג עוד נפרד כפי מה שתראה אותך הבחינה | ||
| + | |- | ||
| + | | | ||
| + | :It is also necessary, in this ratio alone, that between each two odd numbers there will be two means of the natural odd numbers that were skipped. | ||
| + | |style="text-align:right;"|וכבר יתחייב גם כן בזה היחס לבד שיהיה בין כל נפרד ונפרד שני אמצעיים מן הנפרד הטבעי כבר עברו | ||
| + | |- | ||
| + | | | ||
| + | ::Such as 5 and 7 of the natural odd numbers, which were skipped, between 3 and 9. | ||
| + | |style="text-align:right;"|כמו ה' וז' אשר כבר עברו מהנפרד הטבעי בין ג' וט‫' | ||
| + | |- | ||
| + | | | ||
| + | :Likewise it is necessary that between each two even numbers there will be two means of the natural even numbers that were skipped. | ||
| + | |style="text-align:right;"|וכן יחוייב גם כן שיהיה בין הזוג שני אמצעיים כבר עברו מהזוג הטבעי | ||
| + | |- | ||
| + | | | ||
| + | ::Such as 8 and 10, which were skipped, between 6 and 12; as well as 14 and 16, which are between 12 and 18. | ||
| + | |style="text-align:right;"|והזוג כמו ח' וי' אשר כבר עברו בין ו' וי"ב וכמו י"ד וי"ו בין י"ב וי"ח | ||
| + | |- | ||
| + | | | ||
| + | *The quadruple ratio is generated by multiplying each of the terms of the natural numbers by 4 and relating the product to the same term. | ||
| + | |style="text-align:right;"|אמנם {{#annot:quadruple|124|If0k}}הכפל הרביעיי{{#annotend:If0k}} אמנם יתחדש מהכאת כל מדרגה ממדרגות המספר הטבעי בד' עוד יוקיש מה שיצא מן ההכאה אל אותה המדרגה | ||
| + | |- | ||
| + | | | ||
| + | *The same for the quintuple ratio | ||
| + | |style="text-align:right;"|וכן החמשיי | ||
| + | |- | ||
| + | | | ||
| + | *And for any of the species of the following multiple ratios that one wishes. | ||
| + | |style="text-align:right;"|ומה שאחריו ממה שתרצה ממיני הכפלים | ||
| + | |- | ||
| + | |Yet, if the multiple is named by an even number, all of its terms are even numbers. | ||
| + | |style="text-align:right;"|אלא שכאשר היה הכפל נקרא למספר הזוג היו כל מדרגותיו זוגות | ||
| + | |- | ||
| + | | | ||
| + | :Such as the quadruple ratio, of which the beginning of the terms is 4; 8; 12; 16. | ||
| + | |style="text-align:right;"|כמו הכפל הרבעיי אשר תחלת מדרגותיו ארבעה עוד ח' י"ב י"ו | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color: blue; text-align:center;" | ||
| + | |- | ||
| + | |9||8||7||6||5||4||3||2||1 | ||
| + | |- | ||
| + | |36||32||28||24||20||16||12||8||4 | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | |ט||ח||ז||ו||ה||ד||ג||ב||א | ||
| + | |- | ||
| + | |לו||לב||כח||כד||כ||יו||יב||ח||ד | ||
| + | |} | ||
| + | |- | ||
| + | |If the multiple is named by an odd number, its terms are an even number then an odd number. | ||
| + | |style="text-align:right;"|ואם היה הכפל נקרא למספר נפרד היו מדרגותיו אחת זוג ואחרת נפרד | ||
| + | |- | ||
| + | | | ||
| + | :Such as the quintuple ratio, of which the beginning of the terms is 5; 10; 15; 20. | ||
| + | |style="text-align:right;"|כמו הכפל החמשיי אשר תחלת מדרגותיו ה' עוד י' עוד ט"ו עוד כ‫' | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color: blue; text-align:center;" | ||
| + | |- | ||
| + | |9||8||7||6||5||4||3||2||1 | ||
| + | |- | ||
| + | |45||40||35||30||25||20||15||10||5 | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | |ט||ח||ז||ו||ה||ד||ג||ב||א | ||
| + | |- | ||
| + | |מה||מ||לה||ל||כה||כ||טו||י||ה | ||
| + | |} | ||
| + | |- | ||
| + | |This species is named by the greater a multiple ratio and by the smaller a submultiple ratio. | ||
| + | |style="text-align:right;"|וראוי שיקרא זה המין מן הגדול בעל הכפלים ומהקטן תחת בעל הכפלים | ||
| + | |- | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ===== The Discussion on the Second Simple Ratio which is the Superparticular Ratio ===== | ||
| + | |||
| + | |style="text-align:right;"|<big>הדבור ביחס השני הפשוט והוא {{#annot:term|125,1659|I8nQ}}יחס המוסיף חלק{{#annotend:I8nQ}}</big> | ||
| + | |- | ||
| + | |After having discussed the multiple ratio, because it is simpler and anterior by nature as explained, its natural successor, which is the superparticular ratio, is discussed: | ||
| + | |style="text-align:right;"|ואחר שכבר הבאנו הדבור על יחס הכפלים אחר שהוא יותר פשוט ויותר קודם בטבע כמו שבארנו נאמ' על אשר ימשך לו {{#annot:term|2574,2576|KEpZ}}המשכות טבעי{{#annotend:KEpZ}} והוא המוסיף חלק | ||
| + | |- | ||
| + | |When comparing the beginning of the numbers, which is 2, with its successor, which is 3, the three is to two as itself and its half.[<math>\scriptstyle{\color{blue}{3=2+\left(\frac{1}{2}\sdot2\right)}}</math>] | ||
| + | |style="text-align:right;"|וזה שאנו כאשר הקשנו בין תחלת המספרים אשר הוא ב' ובין המספר אשר ימשך לו והוא ג' היה ג' אצל ב' כמהו וכמו חציו | ||
| + | |- | ||
| + | |Hence, the superparticular ratio is formed by comparing the beginning of the numbers with its successor. | ||
| + | |style="text-align:right;"|הנה יתחדש ה{{#annot:term|125,1659|1VcM}}מוסיף חלק{{#annotend:1VcM}} מההקשה בין תחלת המספרים ואשר ימשך לו | ||
| + | |- | ||
| + | | | ||
| + | *the first superparticular ratio by nature: sesquialter ratio | ||
| + | |style="text-align:right;"|והתבאר שהחלק הראשון בטבע הוא {{#annot:sesquialter|125|LmLw}}המוסיף חצי{{#annotend:LmLw}} | ||
| + | |- | ||
| + | | | ||
| + | *sesquitertian ratio: the ratio of the third number, which is 4, to the second number, which is 3 <math>\scriptstyle{\color{blue}{4=3+\left(\frac{1}{3}\sdot3\right)}}</math> | ||
| + | |style="text-align:right;"|עוד {{#annot:sesquitertian|125|40Fb}}המוסיף שליש {{#annotend:40Fb}}בהקשת המספר השלישי אשר הוא ד' אל המספר השני אשר הוא ג' כי ד' כמו ג' וכמו שלישיתו | ||
| + | |- | ||
| + | | | ||
| + | *sesquiquartan ratio | ||
| + | |style="text-align:right;"|עוד {{#annot:sesquiquartan|125|2VLx}}המוסיף רביע{{#annotend:2VLx}} | ||
| + | |- | ||
| + | | | ||
| + | *sesquiquintan ratio | ||
| + | |style="text-align:right;"|עוד {{#annot:sesquiquintan|125|KpVu}}המוסיף חומש{{#annotend:KpVu}} | ||
| + | |- | ||
| + | |the ratio of each number to its predecessor among the natural numbers | ||
| + | |style="text-align:right;"|בהקשת כל מספר אל אשר לפניו מהמספרים הטבעיים | ||
| + | |- | ||
| + | | | ||
| + | *Hence, it is necessary to mention the sesquialter ratio: | ||
| + | |style="text-align:right;"|הנה אם כן יחוייב שנזכיר {{#annot:sesquialter|125|Gy0u}}המוסיף חלק חצי{{#annotend:Gy0u}} | ||
| + | |- | ||
| + | | | ||
| + | :*This ratio is produced by setting the natural even numbers beginning from 2 in a line successively, which is the line that is produced by the double ratio. | ||
| + | |style="text-align:right;"|ונאמר שזה היחס יתילד בשנסדר הזוג הטבעי אשר התחלתו ב' והוא הטור אשר נעשה בכפל השניי בטור ימשך קצתו לקצת | ||
| + | |- | ||
| + | | | ||
| + | :*Setting the numbers that result from the multiplication of each of the terms of the natural numbers by three in a line successively, which is seen in the triple ratio. | ||
| + | |style="text-align:right;"|עוד נסדר המספרים אשר מהכאת מדרגות המספרים הטבעיי' כל א' בג' והוא אשר נראה ב{{#annot:triple ratio|124|IG0u}}כפל השלישיי{{#annotend:IG0u}} בטור ימשכו קצתם לקצת | ||
| + | |- | ||
| + | | | ||
| + | :*Then each term of this line is related to its corresponding in the other line, so the terms illustrate the sesquialter ratio, as seen in the following diagram: | ||
| + | |style="text-align:right;"|עוד יוקש בין כל מדרגה מזה הטור ובין דומה לה מהטור האחר במספר המדרגות כי יראו המדרגות {{#annot:sesquialter|125|Dc37}}יחס המוסיף חלק החציי{{#annotend:Dc37}} כמו שתראה בזאת הצורה | ||
| + | |} | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: auto; text-align:center;" | ||
| + | |- | ||
| + | |לב||ל||כח||כו||כד||כב||כ||יח||יו||יד||יב||י||ח||ו||ד||ב | ||
| + | |- | ||
| + | |מח||מה||מב||לט||לו||לג||ל||כז||כד||כא||יח||טו||יב||ט||ו||ג | ||
| + | |} | ||
| + | |||
| + | {|class="wikitable" style="margin-left: auto; margin-right: auto; color:blue; text-align:center;" | ||
| + | |- | ||
| + | |32||30||28||26||24||22||20||18||16||14||12||10||8||6||4||2 | ||
| + | |- | ||
| + | |48||45||42||39||36||33||30||27||24||21||18||15||12||9||6||3 | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | *The sesquitertian ratio is generated by setting this line of the multiplication of each of the terms of the natural numbers by three parallel to the line that is generated from the multiplication of the natural numbers by four, which is the line that is produced by the quadruple ratio. | ||
| + | |style="text-align:right;"|ואמנם המוסיף שליש הוא אמנם יתחדש בסדור זה הטור אשר מהכאת מדרגות המספרים הטבעיים בג' נכחיי לסדר הטור אשר יתחדש מהכאת המספרים הטבעיים בד' והוא הטור אשר חודש בכפל הרבעיי | ||
| + | |- | ||
| + | | | ||
| + | *Likewise the sesquiquintan ratio, for one of its lines is the line of the quadruple ratio and the other is the line of the quintuple ratio. | ||
| + | |style="text-align:right;"|וכמו כן המוסיף רביע כי אחד מטוריו טור הכפל הרבעיי והאחר טור החמשיי | ||
| + | |- | ||
| + | |The same for any superparticular ratio desired, according to the example described. | ||
| + | |style="text-align:right;"|וכמו כן כל מה שתרצה מהמוסיף חלק הוא כמו זה המשל אשר תארתי לך | ||
| + | |- | ||
| + | |The greater related term in this species is called superparticular and the smaller subsuperparticular. | ||
| + | |style="text-align:right;"|וכבר יתכן שיקרא המוקש בזה המין אם הגדול המוסיף חלק ואם הקטן תחת המוסיף חלק | ||
| + | |- | ||
| + | |So, for any species the smaller related term is called "sub" the specific name of the greater related term above it. | ||
| + | |style="text-align:right;"|וכמו כן יקרא המוקש הקטן מכל מין כאשר הוא תחת מה שלמעלה ממנו מההצטרפות הגדול הנקרא בשם מיוחד | ||
| + | |- | ||
| + | |The discussed multiple and superparticular ratios and their proper enunciation are also noted in this diagram: | ||
| + | |style="text-align:right;"|וכבר רשמתי מה שזכרתי לך מהכפלים והמוסיף חלק ומה שיאות לומר גם בזאת הצורה | ||
| + | |- | ||
| + | | | ||
| + | :{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | |10||9||8||7||6||5||4||3||2||1 | ||
| + | |- | ||
| + | |20||18||16||14||12||10||8||6||4||2 | ||
| + | |- | ||
| + | |30||27||24||21||18||15||12||9||6||3 | ||
| + | |- | ||
| + | |40||36||32||28||24||20||16||12||8||4 | ||
| + | |- | ||
| + | |50||45||40||35||30||25||20||15||10||5 | ||
| + | |- | ||
| + | |60||54||48||42||36||30||24||18||12||6 | ||
| + | |- | ||
| + | |70||63||56||47||42||35||28||21||14||7 | ||
| + | |- | ||
| + | |80||72||64||56||48||40||32||24||16||8 | ||
| + | |- | ||
| + | |90||81||72||63||54||45||36||27||18||9 | ||
| + | |- | ||
| + | |100||90||80||70||60||50||40||30||20||10 | ||
| + | |} | ||
| + | |||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | |י||ט||ח||ז||ו||ה||ד||ג||ב||א | ||
| + | |- | ||
| + | |כ||יח||יו||יד||יב||י||ח||ו||ד||ב | ||
| + | |- | ||
| + | |ל||כז||כד||כא||יח||טו||יב||ט||ו||ג | ||
| + | |- | ||
| + | |מ||לו||לב||כח||כד||כ||יו||יב||ח||ד | ||
| + | |- | ||
| + | |נ||מה||מ||לה||ל||כה||כ||טו||י||ה | ||
| + | |- | ||
| + | |ס||נד||מח||מב||לו||ל||כד||יח||יב||ו | ||
| + | |- | ||
| + | |ע||סג||נו||מז||מב||לה||כח||כא||יד||ז | ||
| + | |- | ||
| + | |פ||עב||סד||נו||מח||מ||לב||כד||יו||ח | ||
| + | |- | ||
| + | |צ||פא||עב||סג||נד||מה||לו||כז||יח||ט | ||
| + | |- | ||
| + | |ק||צ||פ||ע||ס||נ||מ||ל||כ||י | ||
| + | |} | ||
| + | |||
| + | |- | ||
| + | | | ||
| + | *[<math>\scriptstyle{\color{blue}{R_1}}</math>]: Starting by arranging the numbers by natural order, from one to ten, in the first row. | ||
| + | |style="text-align:right;"|וזה שאנו נתחיל ונסדר בטור הראשון הלוקח ברחב המספרים על סדר הטבע מא' עד עשרה | ||
| + | |- | ||
| + | | | ||
| + | *[<math>\scriptstyle{\color{blue}{R_2}}</math>]: The double ratio in the second row, adjacent to the first row. | ||
| + | |style="text-align:right;"|ובטור השני הכפל השני נלוה לטור הראשון | ||
| + | |- | ||
| + | | | ||
| + | *[<math>\scriptstyle{\color{blue}{R_3}}</math>]: The triple ratio in the third row. | ||
| + | |style="text-align:right;"|ובטור השלישי הכפל השלישי | ||
| + | |- | ||
| + | | | ||
| + | *[<math>\scriptstyle{\color{blue}{R_4}}</math>]: The quadruple ratio in the fourth row. | ||
| + | |style="text-align:right;"|ובטור הרביעי הכפל הרביעי | ||
| + | |- | ||
| + | | | ||
| + | *So on, in ten rows, ending with the decuple ratio. [<math>\scriptstyle{\color{blue}{R_{10}}}</math>] | ||
| + | |style="text-align:right;"|וכן עשרה טורים ויהיה הכפל העשריי סופם | ||
| + | |- | ||
| + | |It is clear in this figure that: | ||
| + | *the two lines [<math>\scriptstyle{\color{blue}{R_1;\;C_1}}</math>] that meet on the square 1 and extend to the surface 1-100 | ||
| + | |style="text-align:right;"|וגלוי בזאת הצורה ששני הטורים אשר יפגשו על מרובע א' ויתרחבו עד שטח א'ק‫' | ||
| + | |- | ||
| + | | | ||
| + | *the two lines [<math>\scriptstyle{\color{blue}{R_2;\;C_2}}</math>] that meet on the square 4 and extend to the surface 1-100 | ||
| + | |style="text-align:right;"|ושני הטורים אשר יפגשו על מרובע ד' ויתרחבו עד שטח א'ק‫' | ||
| + | |- | ||
| + | | | ||
| + | *the two lines [<math>\scriptstyle{\color{blue}{R_3;\;C_3}}</math>] that meet on the square 9 and extend to the surface 1-100 | ||
| + | |style="text-align:right;"|ושני הטורים אשר יפגשו על מרובע ט' ויתרחבו עד שטח א'ק‫' | ||
| + | |- | ||
| + | |Their ratio is one, i.e. the ratio of each term to its precedecessor in either of the two lines that meet on one of the squares that are on the diagonal, i.e. the main diagonal of the whole figure, is as the ratio of its corresponding term on the other line to its respective predecessor. | ||
| + | |style="text-align:right;"|יחסם אחד ר"ל ששעור כל מדרגה מאשר לפניה מאחד מכל שני טורים מהם יפגשו על מרובע מן המרובעים אשר על הקוטר ר"ל קוטר הצורה הגדולה כשעור המדרגה אשר היא דומה לה מהטור האחר מאשר היא גם כן לפניה | ||
| + | |- | ||
| + | |Except that: | ||
| + | *Each term of the two first lines [<math>\scriptstyle{\color{blue}{R_1;\;C_1}}</math>] adds one to its neighbor. | ||
| + | |style="text-align:right;"|אלא ששני הטורים הראשונים תוסיף כל מדרגה מהם על אשר ילוה לה מכל אחד משני הטורים אחד | ||
| + | |- | ||
| + | | | ||
| + | *Each term of the two second lines [<math>\scriptstyle{\color{blue}{R_2;\;C_2}}</math>] adds two to its neighbor. | ||
| + | |style="text-align:right;"|ושני הטורים השניים תוסיף כל אחת ממדרגותיהם על אשר תלוה לה שנים שנים | ||
| + | |- | ||
| + | | | ||
| + | *Each term of the two third lines [<math>\scriptstyle{\color{blue}{R_3;\;C_3}}</math>] adds three to its neighbor. | ||
| + | |style="text-align:right;"|ושני הטורים השלישיים תוסיף כל אחת ממדרגותיהם על אשר תלוה שלשה שלשה | ||
| + | |- | ||
| + | | | ||
| + | *The fourth [<math>\scriptstyle{\color{blue}{R_4;\;C_4}}</math>] by four. | ||
| + | |style="text-align:right;"|והרביעיים ארבעה ארבעה | ||
| + | |- | ||
| + | |The ratios of the terms of these lines, [namely] the ratio of any desired term to its predecessor, is one and the same, as they are similar. | ||
| + | |style="text-align:right;"|ויחסי המדרגות מאלו הטורים יחס כל מדרגה אל אשר לפניה שתרצה אחד אחר שיהיו דומים | ||
| + | |- | ||
| + | |For example: | ||
| + | *the ratio of 42 on the seventh row [<math>\scriptstyle{\color{blue}{R_7}}</math>] to its precedecessor 36 on this row is as the ratio of 7 on the first row [<math>\scriptstyle{\color{blue}{R_1}}</math>] to its predecessor 6 [<math>\scriptstyle{\color{blue}{42:36=7:6}}</math>] | ||
| + | |style="text-align:right;"|משל זה שיחס מ"ב מהטור השביעי הלוקח ברחב אל ל"ו אשר לפניה מזה הטור כיחס ז' מהטור הראשון הלוקח ברחב אל ו' אשר לפניו | ||
| + | |- | ||
| + | | | ||
| + | *the ratio of 7 on the first column [<math>\scriptstyle{\color{blue}{C_1}}</math>] to its preceding 6 on this column is as the ratio of 42 on the seventh column [<math>\scriptstyle{\color{blue}{C_7}}</math>] to its predecessor 36 on the same column [<math>\scriptstyle{\color{blue}{7:6=42:36}}</math>] | ||
| + | |style="text-align:right;"|ועוד יחס ז' מהטור הראשון הלוקח באורך אל ו' אשר לפניו בזה הטור כיחס מ"ב מהטור הז' הלוקח באורך אל ל"ו אשר לפניו מאותו הטור | ||
| + | |- | ||
| + | | | ||
| + | :Since the column that goes from 6 on the first row [<math>\scriptstyle{\color{blue}{C_6}}</math>] and the row that goes from 6 on the first column [<math>\scriptstyle{\color{blue}{R_6}}</math>] meet on the square 36, and 36 is the term related to each of the terms 42 on both cross-lines, as both lines intersect on the square 36. | ||
| + | |style="text-align:right;"|לפי שהטור אשר יצא מו' מהטור הראשון ההולך ברחב הולך באורך והטור אשר יצא מו' מהטור הראשון ההולך באורך הולך ברחב יפגשו על מרובע ל"ו והיה ל"ו לכל אחת משתי המדרגות אשר בכל אחת מהן מ"ב משני הטורים המתחלפים מדרגה ראשונה לפי ששני הטורים יתחתכו על מרובע ל"ו | ||
| + | |- | ||
| + | | | ||
| + | :Likewise, each cross row and column [<math>\scriptstyle{\color{blue}{R_n;\;C_n}}</math>] meet on a square among the squares on the main diagonal of the great square, and the square on which they meet is common to both. | ||
| + | |style="text-align:right;"|וכן כל שני טורים מתחלפים באורך וברחב יפגשו על מרובע מן המרובעים אשר על קוטר המרובע הגדול הנה המרובע אשר יפגשו עליו משותף להם | ||
| + | |- | ||
| + | |Hence, it is already clarified how the multiple ratio is produced from its related term: | ||
| + | |style="text-align:right;"|הנה אם כן כבר התבאר איך יתילד בעל הכפלים למה שיוקש אליו | ||
| + | |- | ||
| + | | | ||
| + | *Since the second line on whichever desired side, row or column, to the first line in the same side is a double ratio. [<math>\scriptstyle{\color{blue}{R_2:R_1;\;C_2:C_1}}</math>] | ||
| + | |style="text-align:right;"|לפי שהטור השני מטורי אי זה משני צדי האורך והרחב שתרצה אל הטור הראשון מאותו הצד כפל שניי | ||
| + | |- | ||
| + | | | ||
| + | *The third to it is triple ratio. [<math>\scriptstyle{\color{blue}{R_3:R_1;\;C_3:C_1}}</math>] | ||
| + | |style="text-align:right;"|והשלישי אליו שלשיי | ||
| + | |- | ||
| + | | | ||
| + | *The fourth to it is quadruple ratio [<math>\scriptstyle{\color{blue}{R_4:R_1;\;C_4:C_1}}</math>] | ||
| + | |style="text-align:right;"|והרביעי אליו רבעיי | ||
| + | |- | ||
| + | |So on always by oeder of the natural succession. | ||
| + | |style="text-align:right;"|וכן תמיד כפי סדר על משך הטבע | ||
| + | |- | ||
| + | |This is the explanation of the multiple ratios in this diagram. | ||
| + | |style="text-align:right;"|וזה הוא המאמר על הכפלים בזאת הצורה | ||
| + | |- | ||
| + | |As for the superparticular ratio: | ||
| + | |style="text-align:right;"|אמנם המוסיף חלק | ||
| + | |- | ||
| + | | | ||
| + | :*The two lines, row and column, that proceed from the two squares of 30 and meet on the square of 9, when their terms are related to the terms of both lines, row and column, that proceed from the two squares of 20 and meet on the square of 4, each term to its consecutive, is in sesquialter ratio [<math>\scriptstyle{\color{blue}{R_3:R_2;\;C_3:C_2}}</math>] | ||
| + | |style="text-align:right;"|הנה שני הטורים שלוקח אחד מהם באורך והאחר ברחב וכבר יצאו משני מרובעי ל' ל' ויפגשו על מרובע ט' כאשר יוחסו מדרגותיהם אל מדרגות שני הטורים הלוקחים באורך והרחב וכבר יצאו משני מרובעי כ' כ' ויפגשו על מרובע ד' כל מדרגה אל אשר ילוה לה תמצא זה ביחס המוסיף חלק החציי | ||
| + | |- | ||
| + | | | ||
| + | :*So the ratio of the rest of the terms of these four lines to their beginnings, i.e. the two terms two and three in the rows and the two terms two and three in the columns. | ||
| + | |style="text-align:right;"|וכן יחס שאר מדרגות אלו הטורים הארבעה אל התחלותיהם ר"ל אל שני בתי ב' וג' משני הטורים הלוקחים באורך ואל שני בתי ב' וג' משני הטורים הלוקחים ברחב | ||
| + | |- | ||
| + | | | ||
| + | *The sesquitertian ratio: | ||
| + | |style="text-align:right;"|ואמנם {{#annot:sesquitertian|125|nS12}}יחס המוסיף חלק השלישי{{#annotend:nS12}} | ||
| + | |- | ||
| + | | | ||
| + | :*The two lines, row and column, that proceed from the two squares of 40 and meet on the square of 16, when their terms are related to the terms of both lines, row and column, that proceed from the two squares of 30 and meet on the square of 9, each term to its preceding in the other line. [<math>\scriptstyle{\color{blue}{R_4:R_3;\;C_4:C_3}}</math>] | ||
| + | |style="text-align:right;"|הנה שני הטורים שלוקח אחד מהם באורך והאחר ברחב וכבר יצאו משני מרובעי מ' מ' ויפגשו על מרובע י"ו כאשר יוחסו מדרגותיהם אל מדרגות שני הטורים הלוקחים באורך והרחב היוצאים משני מרובעי ל' ל' ויפגשו על מרובע ט' כל מדרגה אשר לפניה מהטור האחר | ||
| + | |- | ||
| + | | | ||
| + | :This is found in sesquitertian ratio, as in the explanation mentioned for the sesquialter ratio. | ||
| + | |style="text-align:right;"|תמצא זה ביחס המוסיף חלק השלישי בכמו אותו הביאור אשר זכרנו כמוסיף חלק החציי | ||
| + | |- | ||
| + | | | ||
| + | *Likewise for the sesquiquartan ratio. | ||
| + | |style="text-align:right;"|וכן {{#annot:sesquiquartan|125|oTdk}}המוסיף חלק הרביעי{{#annotend:oTdk}} | ||
| + | |- | ||
| + | | | ||
| + | *Likewise for sesquiquintan ratio. | ||
| + | |style="text-align:right;"|וכן {{#annot:sesquiquintan|125|KZkH}}המוסיף חלק החמשיי{{#annotend:KZkH}} | ||
| + | |- | ||
| + | |The species of the superparticular ratio that follow in this art, derive their names from the sequence of the natural numbers that produced from the addition of the units. | ||
| + | |style="text-align:right;"|ומה שאחר זה ממיני המוסיף חלק תמצאהו בזאת המלאכה נמשך בגזרת שמו מ{{#annot:term|2576,2575|jubr}}משך המספרים הטבעיים{{#annotend:jubr}} המתילדים מ{{#annot:term|154,2511|Mdou}}הוספת{{#annotend:Mdou}} האחדים | ||
| + | |- | ||
| + | |Hence it is already clarified to the perception that the most anterior among these ratios, by nature rather than by will and assumption, is the multiple ratio, due to what was ascribed to it. | ||
| + | |style="text-align:right;"|הנה אם כן כבר התבאר לחוש מהיותר קודם באלו היחסים בטבע לא ברצון והנחה בעל הכפלים למה שיוחס אליו | ||
| + | |- | ||
| + | |And the most anterior among [the multiple ratios] is the double ratio, then the triple ratio, and so on always according to the descript derivation of the numbers as they are in the natural order. | ||
| + | |style="text-align:right;"|ושהקודם יותר בזה השניי עוד השלישי וכן תמיד כפי תואר הגזר המספרים כמו שהם בסדר הטבע | ||
| + | |- | ||
| + | |The superparticular ratio then follows the multiple ratio | ||
| + | |style="text-align:right;"|ושאשר ימשך לבעל הכפלים הוא המוסיף חלק | ||
| + | |- | ||
| + | |And the beginning of the [superparticular ratios] is the sesquialter ratio, then the sesquitertian ratio, then the sesquiquartan ratio and so on always according to the derivation of the numbers as they are in the natural order. | ||
| + | |style="text-align:right;"|ושתחלת זה הוא החציי עוד השלישי ועוד הרביעי עוד כן תמיד כפי הגזרה מהמספרים כמו שהם בסדר הטבע | ||
| + | |- | ||
| + | |What can be apprehended from this diagram is that the terms of the numbers on the main diagonal that goes out from 1 to its opposite, 100, each one is rooted, i.e. has an expressible root, which when multiplied by itself, i.e. by the measure of its units, it is equal to the total. [<math>\scriptstyle{\color{blue}{R_n;\;C_n}}</math>] | ||
| + | |style="text-align:right;"|וממה שישיג סדר זאת הצורה שמדרגות המספרים אשר על קוטר היוצא מא' אל ק' הכתוב נגדו כל אחת נגזרת ר"ל שיש לו שורש ו{{#annot:term|1075,2193|VuXT}}ידובר בו{{#annotend:VuXT}} בשיכפל בעצמו ר"ל בשעור אחדיו היה שוה לכלם | ||
| + | |- | ||
| + | | | ||
| + | :Such as: 1; 4; 9; 16; 25; 36; 49; 64; 81; 100 | ||
| + | |style="text-align:right;"|כמו א' ד' ט' י"ו כ"ה ל"ו מ"ט ס"ד פ"א ק‫' | ||
| + | |- | ||
| + | | | ||
| + | :For each of these is a rooted number in actu. | ||
| + | |style="text-align:right;"|כי כל אחד מאלו מספר נגזר בפעל | ||
| + | |- | ||
| + | | | ||
| + | :*Except for the one, which is rooted in potentia and its root is one, as it is one itself. <math>\scriptstyle{\color{blue}{\sqrt{1}=1}}</math> | ||
| + | |style="text-align:right;"|מלבד האחד שהוא נגזר בכח וגדרו אחד לפי שהוא אחד בעצמו | ||
| + | |- | ||
| + | | | ||
| + | :*<math>\scriptstyle{\color{blue}{\sqrt{4}=2}}</math> | ||
| + | |style="text-align:right;"|וגדר ד' ב‫' | ||
| + | |- | ||
| + | | | ||
| + | :*<math>\scriptstyle{\color{blue}{\sqrt{9}=3}}</math> | ||
| + | |style="text-align:right;"|וגדר ט' ג‫' | ||
| + | |- | ||
| + | |If the roots of these terms are taken and arranged in a line, they are found in the order of their terms, by the order of the sequence of the natural numbers, such as 1, 2, 3, 4, 5, 6, until the end of the rooted numbers that were set on the main diagonal of the square. | ||
| + | |style="text-align:right;"|ואם לקחת גדרי אלו המדרגות ותסדרם בטור תמצאם בסדר מדרגותיהם {{#annot:term|2576,2575|5ppE}}על סדר משך המספרים הטבעיים{{#annotend:5ppE}} כמו א' ב' ג' ד' ה' ו' עד תכלית מה שהנחת מן המספרים הנגדרים באלכסון המרובע | ||
| + | |- | ||
| + | |'''Abū Yusūf''' '''said''': we have found these rooted numbers produced and grow from the sum of the natural odd numbers to each other by their successive order, beginning from the one, which is an odd number in potantia. | ||
| + | |style="text-align:right;"|<big>אמר אבו יוסף</big> מצאנו אלו המספרים הנגדרים אמנם יתחדשו ויצמחו מתוספת הנפרדים הטבעיים על משך סדרם אשר התחלתם מהאחד אשר הוא נפרד בכח על קצתם על קצת | ||
| + | |- | ||
| + | | | ||
| + | :*<math>\scriptstyle{\color{blue}{1^2+\left(1+2\right)=1+3=4=2^2}}</math> | ||
| + | |style="text-align:right;"|ג' כאשר נוסף על האחד יתקבץ מספר נגדר והוא ד' וגדרו ב‫' | ||
| + | |- | ||
| + | | | ||
| + | :*<math>\scriptstyle{\color{blue}{2^2+\left(3+2\right)=4+5=9=3^2}}</math> | ||
| + | |style="text-align:right;"|וכאשר נוסף על ד' הנפרד אשר ימשך לג' והוא ה' יתקבץ מספר נגדר והוא ט' וגדרו ג‫' | ||
| + | |- | ||
| + | | | ||
| + | :*<math>\scriptstyle{\color{blue}{3^2+\left(5+2\right)=9+7=16=4^2}}</math> | ||
| + | |style="text-align:right;"|וכאשר נוסף הנפרד אשר ימשך לה' והוא ז' על ט' יתקבץ מספר נגדר והוא י"ו ושרשו ד‫' | ||
| + | |- | ||
| + | | | ||
| + | :*and so on always. | ||
| + | |style="text-align:right;"|וכן תמיד הנה | ||
| + | |- | ||
| + | |Thus, it is clarified that when the natural odd numbers, beginning from the one, which is odd in potentia, are added to each other by their successive order, the natural rooted numbers are produced by their successive order. | ||
| + | |style="text-align:right;"|אם כן כבר התבאר שכאשר נוספו הנפרדים הטבעיים אשר התחלתם האחד אשר הוא נפרד בכח קצתם על קצת על משך סדרם יתילדו המספרים הנגדרים הטבעיים על משך סדרם | ||
| + | |- | ||
| + | |Their roots that are extracted from them are by their successive order as the natural numbers | ||
| + | |style="text-align:right;"|והיו שרשיהם לקוחים מהם על משך סדרם על המספר הטבעי | ||
| + | |- | ||
| + | |The terms of the two secondary diagonals that follow the main diagonal are heteromecic, i.e. the two numbers that the term is a product of their multiplication one by the other - one of them exceeds the other by one, as they are conceived as close to the root of the term that has an expressible root. | ||
| + | |style="text-align:right;"|ואמנם שני הטורים אשר ימשכו וילוו לקוטר הנה מדרגותיהם {{#annot:term|1772,2115|l7zm}}זולתיות האורך{{#annotend:l7zm}} ר"ל ששני המספרים אשר התקבצה המדרגה מהכאת אחד מהם באחר יוסיף אחד מהם על האחר באחד בשיודמו שכבר קרבו מגדר המדרגה אשר היה להם {{#annot:term|1075,2193|7pvI}}גדר מספר ידובר בו{{#annotend:7pvI}} | ||
| + | |- | ||
| + | | | ||
| + | :*<math>\scriptstyle{\color{blue}{6=2\times3}}</math> | ||
| + | |style="text-align:right;"|ו{{#annot:term|1772,2198|kY9X}}המספרים הזולתיים{{#annotend:kY9X}} כמו ו' כי הוא מהכאת ב' בג‫' | ||
| + | |- | ||
| + | | | ||
| + | :*<math>\scriptstyle{\color{blue}{12=3\times4}}</math> | ||
| + | |style="text-align:right;"|וכמו י"ב שהוא מהכאת ג' בד‫' | ||
| + | |- | ||
| + | |So they are always found in the two secondary diagonals that are on both sides of the main diagonal. | ||
| + | |style="text-align:right;"|עוד כן נמצאם תמיד בשני הטורים אשר משני צדי הקוטר כלם | ||
| + | |- | ||
| + | |What can be apprehended from this diagram is that the two lines that go out from the square of 1, lengthwise and breadthwise - their terms are added one by one until each of them reaches to ten that is the end of all their terms. [<math>\scriptstyle{\color{blue}{R_1;\;C_1}}</math>] | ||
| + | |style="text-align:right;"|וממה שישיג זאת הצורה ששני הטורים היוצאים ממרובע א' באורך והרחב יתוספו מדרגותיהם אחד אחד עד שיגיע כל אחד מהם אל העשרה אשר הם סוף כל המדרגות בם | ||
| + | |- | ||
| + | |And that the two lines that go out from the squares [i.e. cells] of 10 and meet on the square of 100 - their terms are added ten by ten. [<math>\scriptstyle{\color{blue}{R_{10};\;C_{10}}}</math>] | ||
| + | |style="text-align:right;"|וששני הטורים היוצאים משני מרובעי י"י ויפגשו על מרובע ק' יתוספו מדרגותיהם י' י‫' | ||
| + | |- | ||
| + | |Furthermore, if what is on the four angles of this diagram is summed, one finds this sum a rooted number. | ||
| + | |style="text-align:right;"|ואם קובץ מה שבזויות זאת הצורה הארבעה תמצא כלל זה המספר נגדר | ||
| + | |- | ||
| + | |What can be apprehended from this diagram is that the sum of the terms of each square whose main diagonal differs from the main diagonal of the entire diagram, is rooted | ||
| + | |style="text-align:right;"|וממה שישיג זאת הצורה שכלל מדרגות כל מרובע יהיה קטרו נבדל מקוטר הצורה הוא נגדר | ||
| + | |- | ||
| + | |'''Abū Yusūf''' '''said''': what is apprehended concerning the other main diagonal, whose ends are ten, and the number of its terms is even, is that it has two means, which are 30. | ||
| + | |style="text-align:right;"|<big>אמר אבו יוסף</big> וממה שישיג הקוטר האחר אשר תכליותיו עשרה עשרה ומספר מדרגותיו זוג שיש לו שני אמצעיים והם ל' ל‫' | ||
| + | |- | ||
| + | |The terms that are between one of the two ends and one of the two means, the ratio between them, and the values of their numbers are in the same ratio of what is between the other end and the other mean. | ||
| + | |style="text-align:right;"|והמדרגות אשר בין אחד משני התכליות עד אחד משני האמצעיים ביחס קצתם אל קצת ו{{#annot:term|217,1675|CcHP}}הגעות מספריהם{{#annotend:CcHP}} כיחס מה שבין התכלית האחר והאמצעי האחר | ||
| + | |- | ||
| + | |'''The philosopher, the author of this book''' [= Nicomachus] '''said''': That which takes place in this diagram is found when its investigation is precise, for it has more beneficial and pure things than was described, but they will not be investigated in this ''introduction'', as there was no intention to investigate them here. | ||
| + | |style="text-align:right;"|<big>אמר הפילוסוף מניח הספר</big> כבר נמצא המקויים בזאת הצורה כאשר ידוקדק העיון בה כי בה דברים מהתועלות והזכיות יותר מאשר תארנו אלא שאנו לא נחקרם בזה המבוא לפי שאין כונתנו בו לחקרם | ||
| + | |- | ||
| + | |It is necessary to move forward to the explanation of what is firmly agreed upon, concerning the account on the five ratios that we started discussing | ||
| + | |style="text-align:right;"|וראוי שנעתק אל המאמר על מה שהוא חזק ההאותות למה שהחלונו לדבר בו מזכר היחסים החמש | ||
| + | |- | ||
| + | |Now, it should be said that the discussion of the superpartient should be anterior, as it is simpler than the two remaining ratios: | ||
| + | |style="text-align:right;"|ונאמר עתה שהמוסיף חלקים למה שהוקש אליו יותר ראשון להקדים המאמר עליו לפי שהוא יותר פשוט משני היחסים הנשארים | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *The multiple superparticular ratio consists of two ratios. |
| + | |style="text-align:right;"|וזה שבעל הכפלים המוסיף חלק הוא מורכב משני יחסים | ||
|- | |- | ||
| | | | ||
| − | === | + | *The multiple superpartient ratio consists of two ratios as well. |
| − | + | |style="text-align:right;"|ובעל הכפלים המוסיף חלקים מורכב גם כן משני יחסים | |
| + | |- | ||
| + | |Furthermore, when the beginning of the odds, which is 3, is added to the second odd number, which is 5, the superbipartient is generated, which is the beginning of the species of the superpartient ratios. | ||
| + | |style="text-align:right;"|ועוד שאנו כאשר חברנו ראש הנפרדים אשר הוא ג' אל הנפרד השני אשר הוא ה' יתחדש {{#annot:superbipartient|128|DyCx}}המוסיף שני חלקים{{#annotend:DyCx}} והוא תחלת מיני המוסיף חלקים | ||
| + | |- | ||
| + | |Therefore, it is necessary to place the discussion of this ratio after what was said about the two first simple ratios. | ||
| + | |style="text-align:right;"|ולזה יחוייב שנשים המאמר על זה היחס נמשך למה שאמרנו משני היחסים הראשונים הפשוטים | ||
| + | |- | ||
| + | |In accordance with the natural order, the multiple superparticular ratio appears before the superpartient ratio. | ||
| + | |style="text-align:right;"|ואמנם בהצטרף על סדר הטבע הנה הכפל המוסיף חלק יראה קודם מהמוסיף חלקים | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :This occurs when we first relate two numbers of the terms of the natural numbers, the relating of which generates a third [i.e. new kind of] ratio. |
| + | |style="text-align:right;"|וזה כשנקיש תחלה שני מספרים ממדרגות המספרים הטבעיים יתחדש בהקשתם יחס שלישי | ||
|- | |- | ||
| | | | ||
| − | === | + | ::Such as, relating 5 to 2, for 5 is the same as double 2 plus its half, and its half is a part of it. <math>\scriptstyle{\color{blue}{5=\left(2\sdot2\right)+\left(\frac{1}{2}\sdot2\right)}}</math> |
| + | |style="text-align:right;"|והוא כמו צרוף ה' אל ב' כי ה' כמו כפל ב' וכמו חציו וחציו חלק ממנו | ||
| + | |- | ||
| + | | | ||
| + | ::and 5:2 are the first two numbers of the terms of the natural numbers, which by the relation of one of them to the other a third ratio is generated. | ||
| + | |style="text-align:right;"|וה' ב' ראשוני שני מספרים ממדרגות המספרים הטבעיים יתחדש בהצטרף אחד מהם אל האחר יחס שלישי | ||
| + | |- | ||
| + | |Yet, it is clear and obvious that the superpartient ratio is simpler than the multiple superparticular ratio. | ||
| + | |style="text-align:right;"|אבל הוא מבואר נגלה שיחס המוסיף חלקים יותר פשוט מיחס הכפל המוסיף חלק | ||
| + | |- | ||
| + | |The simple is anterior to the compound by natural precedence. | ||
| + | |style="text-align:right;"|והפשוט יותר קודם מהמורכב קדימה טבעית | ||
| + | |- | ||
| + | |Hence, it is necessary to preface the discussion on the superpartient. | ||
| + | |style="text-align:right;"|הנה אם כן יחוייב שנקדים המאמר על המוסיף חלקים אל מה שיוקש אליו | ||
| + | |- | ||
| + | |And among its species, the superbipartient is first, since it is the first of its species. | ||
| + | |style="text-align:right;"|ונקדים ממיניו המוסיף שני חלקים אחר שהוא ראש מיניו | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ===== The Discussion of the Third Ratio which is the Superpartient Ratio ===== | ||
| − | + | |style="text-align:right;"|<big>הדבור ביחס השלישי והוא היחס המוסיף חלקים</big> | |
| + | |- | ||
| + | |'''Nicomachus''' '''said''': there is no superpartient ratio less than the superbipartient ratio | ||
| + | |style="text-align:right;"|<big>אמר ניקומאכוש</big> לא יהיה {{#annot:term|128,1660|ZX2O}}היחס המוסיף חלקים{{#annotend:ZX2O}} בפחות מהמוסיף שני חלקים | ||
| + | |- | ||
| + | |Hence, this ratio is formed from the sequence of the natural numbers beginning with three in a line. | ||
| + | |style="text-align:right;"|ולזה אמנם יתילד זה היחס מסדר המספרים הטבעיים אשר התחלתם השלשה בטור | ||
| + | |- | ||
| + | |Because the number prior to three has no two parts that are smaller than it, since its parts are equal to it.[?] | ||
| + | |style="text-align:right;"|לפי שהמספר אשר קודם השלשה אין לו שני חלקים יהיו פחות ממנו אחר שחלקיו שוים לו | ||
| + | |- | ||
| + | |He said: the terms of the natural odd numbers are arranged beneath the terms of the mentioned line, beginning with the successor of 3, which is 5. | ||
| + | |style="text-align:right;"|<big>אמר</big> ויסודר תחת מדרגות הטור הנזכר מדרגות הנפרדים הטבעיים אשר הראשון מהם ימשך לג' והוא ה‫' | ||
| + | |- | ||
| + | |Then, each term is related to the one above it. | ||
| + | |style="text-align:right;"|עוד נקיש בין כל מדרגה ואשר למעלה ממנו | ||
| + | |- | ||
| + | |Thus, all successive species of the superpartient ratio are revealed, according to the rule of their succession in nature. | ||
| + | |style="text-align:right;"|ויראו לנו כל מיני {{#annot:term|128,1660|DE29}}המוסיף חלקים{{#annotend:DE29}} נמשכים כפי משפט המשכם בטבע | ||
| + | |- | ||
| + | |If one wishes to see the production of each species of this ratio: | ||
| + | |style="text-align:right;"|ואמנם כאשר תרצה שתראה איך צמיחת כל מין ממיני זה היחס | ||
| + | |- | ||
| + | | | ||
| + | *Placing the two first numbers that produce that species in one position, the smaller above the greater. | ||
| + | |style="text-align:right;"|הנה עתה תעמיד שני המספרים הראשונים הפועלים למין ההוא במדרגה אחת ותניח הקטן למעלה מהגדול | ||
| + | |- | ||
| + | | | ||
| + | *Multiplying each by 2, then placing the two products in the successive position after the two first numbers, each follows its counterpart, the greater follows the greater and the smaller follows the smaller. | ||
| + | |style="text-align:right;"|עוד תכה כל אחד מהם בב' ותעמיד שני המספרים המגיעים אחר ההכאה במדרגה תמשך לשני המספרים הראשונים כל אחת מהן תמשך לדומה לה הגדול ימשך לגדול והקטן ימשך לקטן | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *Multiplying again each of the first two numbers by 3, then placing the [products] in the third successive position, as the two numbers were placed in the second position. |
| + | |style="text-align:right;"|עוד תשוב אל שני המספרים הראשונים ותכה אותה גם כן בג' ותעמידם במדרגה שלישית על הצד אשר העמדת בו שני המספרים במדרגה השנית | ||
|- | |- | ||
| | | | ||
| − | ==== | + | *Multiplying each of the first two numbers also by 4, then placing the [products] according the above concept in the position denominated by the number that multiplied the first two numbers. |
| + | |style="text-align:right;"|וכן גם כן תכה שני המספרים הראשונים בד' ותעמידם כפי הגדר הנזכר במדרגה הנגזרת השם מהמספר אשר הכית שני המספרים הראשונים בו | ||
| + | |- | ||
| + | |The same is done always when wishing to yield any species of the superpartient ratio. | ||
| + | |style="text-align:right;"|וכן תעשה תמיד כאשר תרצה להצמיח מין ממיני המוסיף חלקים | ||
| + | |- | ||
| + | |Setting up the first numbers that produce each species of the superpartient ratio by the order of these natural species, so that no species of them would fall in the vacuum of the given numbers - it is as mentioned in the introduction of this chapter, and as the first numbers that produce the species of the superpartient ratio that are illustrated below. | ||
| + | |style="text-align:right;"|ואמנם איך תניח המספרים הראשונים הפועלים למיני המוסיף חלקים על סדר אותם המינים הטבעיים עד שלא יפול מהם מין בפנוי מה שיונח מהמספרים הנה הוא כמו שנזכר בפתיחת זה הפרק וכמו שכבר המשלנו תחת זה המספרים הראשונים הפועלים למיני {{#annot:term|128,1660|29Ia}}יחס המוסיף חלקים{{#annotend:29Ia}} | ||
| + | |- | ||
| + | |This is the diagram: | ||
| + | |style="text-align:right;"|וזאת הצורה ועד כאן דברי מחבר הספר | ||
| + | |} | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: auto; text-align:center;" | ||
| + | |- | ||
| + | |יט||יח||יז||יו||טו||יד||יג||יב||יא||י||ט||ח||ז||ו||ה||ד||ג | ||
| + | |- | ||
| + | |לז||לה||לג||לא||כט||כז||כה||כג||כא||יט||יז||טו||יג||יא||ט||ז||ה | ||
| + | |} | ||
| + | |||
| + | {|class="wikitable" style="color:blue; margin-left: auto; margin-right: auto; text-align:center;" | ||
| + | |- | ||
| + | |19||18||17||16||15||14||13||12||11||10||9||8||7||6||5||4||3 | ||
| + | |- | ||
| + | |37||35||33||31||29||27||25||23||21||19||17||15||13||11||9||7||5 | ||
| + | |} | ||
| + | |||
| + | {| | ||
| + | |- | ||
| + | |superbitertian ratio | ||
| + | {|class="wikitable" style="color: blue; text-align:center;" | ||
| + | |- | ||
| + | |24||21||18||15||12||9||6||3 | ||
| + | |- | ||
| + | |40||35||30||25||20||15||10||5 | ||
| + | |} | ||
| + | |style="text-align:right;"|מוסיף ב' שלישיות | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | |כד||כא||יח||טו||יב||ט||ו||ג | ||
| + | |- | ||
| + | |מ||לה||ל||כה||כ||טו||י||ה | ||
| + | |} | ||
| + | |- | ||
| + | |supertriquartan ratio | ||
| + | {|class="wikitable" style="color: blue; text-align:center;" | ||
| + | |- | ||
| + | |9||8||7||6||5||4||3||2||1 | ||
| + | |- | ||
| + | |45||40||35||30||25||20||15||10||5 | ||
| + | |} | ||
| + | |style="text-align:right;"|מוסיף ג' רביעיות | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | |לב||כח||כד||כ||יו||יב||ח||ד | ||
| + | |- | ||
| + | |נו||מט||מב||לה||כח||כא||יד||ז | ||
| + | |} | ||
| + | |- | ||
| + | |Until here the author's words. | ||
| + | |style="text-align:right;"|ועד כאן דברי מחבר הספר | ||
| + | |- | ||
| + | !How the other species are extracted, which are similar to whichever of these first species that one wishes: | ||
| + | |style="text-align:right;"|אמנם איך יוצאו שאר המינים הדומים באי זה מין שנרצה מאלו המינים הראשונים | ||
| + | |- | ||
| + | | | ||
| + | :Such as, for example, the superbitertian ratio under the superbiquintan ratio, the superbiseptian ratio, and the rest of what falls under the superbipartient ratios. | ||
| + | |style="text-align:right;"|כמו הדומה דרך משל ב{{#annot:superpartient|128|6vkL}}מוסיף שני שלישים{{#annotend:6vkL}} תחת {{#annot:superbiquintan|128|pCVd}}המוסיף שני חומשים{{#annotend:pCVd}} ושני שביעים ושאר מה שיפל תחת המוסיף שני חלקים | ||
| + | |- | ||
| + | | | ||
| + | :Or those that are similar by falling under the supertripartient ratios. | ||
| + | |style="text-align:right;"|או במתדמים לנפלם תחת {{#annot:supertripartient|128|RzOH}}המוסיפים שלשה חלקים{{#annotend:RzOH}} | ||
| + | |- | ||
| + | | | ||
| + | :Or whichever of the species of the superpartient ratios other than these two. | ||
| + | |style="text-align:right;"|או בלתי שני אלו מאי זה ממיני המוסיף חלקים | ||
| + | |- | ||
| + | |The obvious way for finding this is by arranging the natural numbers in a line, such that the first of its terms is the number that is the number of the additional parts of the species intended to be added to its extracted similar. | ||
| + | |style="text-align:right;"|הנה הדרך הגלוי אל מציאות זה הוא בסדור המספרים הטבעיים בטור יהיה ראש מדרגותיו המספר אשר הוא מספר החלקים הנוספים במין המכוון להוסיף להוצאת הדומים לו | ||
| + | |- | ||
| + | | | ||
| + | :*The first term is added to the second, and the sum is placed under the second. | ||
| + | |style="text-align:right;"|ותנשא המדרגה הראשונה על השנית ויונח מה שיתקבץ תחת השנית | ||
| + | |- | ||
| + | | | ||
| + | :*The first term is [summed] also with the third, [and the sum is placed] under the third | ||
| + | |style="text-align:right;"|עוד נניח המדרגה הראשונה גם כן עם השלישית תחת השלישית | ||
| + | |- | ||
| + | | | ||
| + | :*Also the first with the fourth placed under the fourth. | ||
| + | |style="text-align:right;"|וכן תונח הראשונה עם הרביעית תחת הרביעית | ||
| + | |- | ||
| + | | | ||
| + | :*Proceeding likewise until the given end of the natural numbers. | ||
| + | |style="text-align:right;"|ונעשה זה תמיד עד תכלית המונח מן המספרים הטבעיים | ||
| + | |- | ||
| + | | | ||
| + | *Then each of the terms is related to its counterpart. | ||
| + | |style="text-align:right;"|עוד תקיש בין כל מדרגה ממנה והדומה לה | ||
| + | |- | ||
| + | |An example is given of the superquadripartient ratio, in order that the mathematician will examine it and will be led straight by it in other [cases]. | ||
| + | |style="text-align:right;"|וכבר הנחתי לזה משל מ{{#annot:superquadripartient|128|KN6P}}המוסיף ארבעה{{#annotend:KN6P}} כדי שיבחנהו החושב ויתישר בו בזולתו | ||
| + | |- | ||
| + | |There are species of superpartient ratio that are included in other species of superpartient ratio: | ||
| + | |style="text-align:right;"|ודע שמהמוסיף חלקים מה שיכנס במין אחר מהמוסיף חלקים | ||
| + | |- | ||
| | | | ||
| + | *6 and 10 are in the superquadrisextan ratio [<math>\scriptstyle{\color{blue}{10:6=1+\frac{4}{6}}}</math>] | ||
| + | |style="text-align:right;"|כמו ו' וי' כי הם בהצטרף המוסיף הארבעה שתויות | ||
| + | |- | ||
| + | | | ||
| + | :they are also in superbitertian ratio [<math>\scriptstyle{\color{blue}{10:6=1+\frac{2}{3}}}</math>] | ||
| + | |style="text-align:right;"|והם גם כן בהצטרף המוסיף שני שלישים | ||
| + | |- | ||
| + | |There are species that are included in a ratio of another genus i.e. the superparticular ratio: | ||
| + | |style="text-align:right;"|וממנו גם כן מה שיכנס בזולת סוגו ר"ל במוסיף חלק | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *8 and 12 are in the superquadrioctan ratio [<math>\scriptstyle{\color{blue}{12:8=1+\frac{4}{8}}}</math>] |
| + | |style="text-align:right;"|כמו ח' וי"ב שהם בהצטרף המוסיף הארבעה שמיניות | ||
|- | |- | ||
| | | | ||
| − | ===== | + | :they are also in a superparticular ratio, i.e. sesquialter ratio [<math>\scriptstyle{\color{blue}{12:8=1+\frac{1}{2}}}</math>] |
| + | |style="text-align:right;"|והם גם כן בצרוף המוסיף חלק ר"ל חצי | ||
| + | |- | ||
| + | |If one wishes to know the absolute ratios that are not included in another species, '''al-Kindī''''s effort to set a special technique for each species is not enough, since he strove for what is impossible to complete. | ||
| + | |style="text-align:right;"|ואם תרצה במין מה להכיר הצרופים הגמורים אשר לא יכנסו בזולת מינם לא יספיק בזה מה שטרח בו אלכנדי מהניח מלאכה מיוחדת לכל מין לפי שהוא השתדל במה שלא נוכל לבא עד תכליתו | ||
| + | |- | ||
| + | |However, the general technique for all that was brought of this is to skip each term of the terms of the natural numbers, whose number is commensurable with the number of the additional parts. | ||
| + | |style="text-align:right;"|אבל המלאכה הכוללת לכל מה שהובא מזה היא שנדלג ממדרגות המספרים הטבעיים כל מדרגה שיהיה מספרה משותף למנין החלקים הנוספים | ||
| + | |- | ||
| + | |The meaning of commensurability is that one of the two numbers is part of the other, or else, only if they have a common divisor other than one that counts both of them. | ||
| + | |style="text-align:right;"|ור"ל בהשתתפות שיהיה אחד משני המספרים חלק לאחר ולא יהיה זה אלא אם להם חלק משותף בלתי האחד ימנם יחד | ||
| + | |- | ||
| + | |Its explanation was already introduced in the account on the even number and the account on the composite odd number. | ||
| + | |style="text-align:right;"|וזה כבר עבר ביאורו בזכירת המספר הזוג וזכירת המספר הנפרד המורכב | ||
| + | |- | ||
| + | |Here, a technique is required for recognizing the terms that should be skipped. | ||
| + | |style="text-align:right;"|ומכאן תבוקש התחבולה בהכרת המדרגות אשר נצטרך הנה לדלגם | ||
| + | |- | ||
| + | |These, as was said, are the terms: either such that ratios of the numbers of the additional parts to their corresponding numbers are in a superparticular ratio, or else, if the numbers of the additional parts are not a part of their corresponding numbers, such that their numbers and the number of the additional parts have a common part that counts them both other than one. | ||
| + | |style="text-align:right;"|והיה כמו שאמרנו המדרגות אשר אם שיהיה מנין החלקים המוסיפים מכל אחד ממספריהם כי צירופי אלו יכנסו במוסיף חלק ואם שלא יהיה מנין החלקים המוסיפים חלק מכל אחד ממספריהם אלו שמספריהם ולמנין החלקים הנוספים חלק משותף להם בלתי האחד ימנה אותם יחד | ||
| + | |- | ||
| + | |For, these ratios, even if they are not included in any other than their genus, i.e. in the superparticular ratio, they are included in a species other than their species within the superpartient ratio. | ||
| + | |style="text-align:right;"|כי צירופי אלו ואם לא יכנסו בבלתי סוגם ר"ל במוסיף חלק הנה יכנסו במין בלתי מינם מהמוסיף חלקים | ||
| + | |- | ||
| + | |Hence, when the terms that are commensurable to the number of the parts are skipped, the terms, whose ratios are not included in another genus, remain, and they are the absolute of the terms of the superpartient ratio. | ||
| + | |style="text-align:right;"|הנה כאשר תדלג אלו המדרגות המשותפות למנין החלקים הנה כבר השארת המדרגות אשר לא יכנסו צרופיהם בבלתי מינם והם הגמורים ממדרגות המוסיף חלקים | ||
| + | |- | ||
| + | |We describe the above example by this diagram, with the marking of the commensurable terms: | ||
| + | |style="text-align:right;"|וכבר תארנו משל זה שאמרנו בזאת הצורה עם רושם השותפים הנזכרים בה | ||
| + | |- | ||
| + | |} | ||
| − | + | {|class="wikitable" style="margin-left: auto; margin-right: auto; text-align:center; font-size:87%;" | |
|- | |- | ||
| + | |<small>שתוף</small>|| ||<small>שתוף</small>|| ||<small>שתוף</small>|| ||<small>שתוף</small>|| ||<small>שתוף</small>|| ||<small>שתוף</small>|| ||<small>שתוף</small>|| ||<small>שתוף</small>|| ||<small>שתוף</small>|| ||<small>שתוף</small>|| ||<small>שתוף</small>|| ||<small>שתוף</small>|| || | ||
| + | |- | ||
| + | |כח||כז||כו||כה||כד||כג||כב||כא||כ||יט||יח||יז||יו||טו||יד||יג||יב||יא||י||ט||ח||ז||ו||ה||ד | ||
| + | |- | ||
| + | |לב||לא||ל||כט||כח||כז||כו||כה||כד||כג||כב||כא||כ||יט||יח||יז||יו||טו||יד||יג||יב||יא||י||ט|| | ||
| + | |} | ||
| + | |||
| + | {|class="wikitable" style="margin-left: auto; margin-right: auto; color:blue; text-align:center; font-size:87%;" | ||
| + | |-style="font-size:60%;" | ||
| + | |<small>common</small>|| ||<small>common</small>|| ||<small>common</small>|| ||<small>common</small>|| ||<small>common</small>|| ||<small>common</small>|| ||<small>common</small>|| ||<small>common</small>|| ||<small>common</small>|| ||<small>common</small>|| ||<small>common</small>|| ||<small>common</small>|| || | ||
| + | |- | ||
| + | |28||27||26||25||24||23||22||21||20||19||18||17||16||15||14||13||12||11||10||9||8||7||6||5||4 | ||
| + | |- | ||
| + | |32||31||30||29||28||27||26||25||24||23||22||21||20||19||18||17||16||15||14||13||12||11||10||9|| | ||
| + | |} | ||
| + | |||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== [Compound Ratios] ==== | ||
| + | |||
| | | | ||
| − | |||
|- | |- | ||
| | | | ||
| − | ===== Superparticular Ratio ===== | + | ===== The Discussion on the Fourth Ratio which is the Multiple Superparticular Ratio ===== |
| − | + | |style="text-align:right;"|<big>הדבור ביחס הרביעי והוא יחס הכפל המוסיף חלק</big> | |
| + | |- | ||
| + | |'''The author of this book''' [= Nicomachus] '''said''': The simple species among the species of the relative quantity are the species of the three ratios of the preceding discussion. | ||
| + | |style="text-align:right;"|<big>אמר מניח הספר</big> אמנם המינים הפשוטים ממיני הכמה המצטרף הנה הם מיני שלשת היחסים אשר קדם המאמר עליהם | ||
| + | |- | ||
| + | |For they are like causes and principles for the two remaining species, i.e. the multiple superparticular ratio and the multiple superpartient ratio. | ||
| + | |style="text-align:right;"|כי הם כהתחלות וכפנות לשני המינים הנשארים ר"ל מין הכפל המוסיף חלק מן הכפלים המוסיף חלקים | ||
| + | |- | ||
| + | |Since these two species are produced from the first species and are absorbed in them. | ||
| + | |style="text-align:right;"|אחר ששני אלו המינים כבר יתילדו מהמינים הראשונים ויותכו אליהם | ||
| + | |- | ||
| + | |The simple has also another aspect, by which the species of the multiple ratio and the superparticular ratio are set aside from the superpartient ratio, in that the first is said to resemble to the second. | ||
| + | |style="text-align:right;"|ולפשוט גם כן צד אחר ייוחד בו מין הכפל ומין המוסיף חלק בלתי מין המוסיף חלקים והוא בשיאמר שהוא יתדמה הראשון ממנו לשני | ||
| + | |- | ||
| + | |It is said to be simple, since its meaning is that the two numbers that relate in each of these two species are assimilated one to the other: | ||
| + | |style="text-align:right;"|ויאמר לו פשוט לפי שעניינו אמנם הוא של שני המספרים המצטרפים בכל אחד מאותם שני המינים התדמות בעצמם | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Either the assimilation of two numbers in the multiple ratio, where the greater is composed from the smaller; |
| + | |style="text-align:right;"|אם התדמות שני המספרים במין הכפל באשר הגדול מחובר מהקטן | ||
|- | |- | ||
| | | | ||
| − | ===== | + | :Or the assimilation of two numbers in the superparticular ratio, where they are both composed from one principle, which is the difference between them. |
| + | |style="text-align:right;"|ואם התדמות שני המספרים במין המוסיף חלק באשר הם יחד מחוברים מפנה אחת והוא המותר אשר ביניהם | ||
| + | |- | ||
| + | |Therefore, the ancients related the natural things by relation of these two species alone, and did not relate them by the third species that is said to be simple as well, i.e. the superpartient ratio. | ||
| + | |style="text-align:right;"|ולזה הקישו הראשונים הדברים הטבעיים החבור בשני אלו המינים לבד ולא יקישו אותם במין השלישי אשר יאמר לו שהוא פשוט גם כן ר"ל במין המוסיף חלקים | ||
| + | |- | ||
| + | |The two compound species: | ||
| + | |style="text-align:right;"|ואמנם שני המינים המורכבים | ||
| + | |- | ||
| + | | | ||
| + | :*One of them consists of the multiple ratio and the superparticular ratio. | ||
| + | |style="text-align:right;"|הנה אחד מהם מורכב מבעל הכפלים ומהמוסיף חלק | ||
| + | |- | ||
| + | | | ||
| + | :*The other consist of the multiple ratio and the superpartient ratio. | ||
| + | |style="text-align:right;"|ואמנם האחר מורכב מבעל הכפלים ומהמוסיף חלקים | ||
| + | |- | ||
| + | |Since the part is anterior by nature to the parts, the multiple superparticular ratio is anterior to the multiple superpartient ratio. | ||
| + | |style="text-align:right;"|ולפי שהחלק קודם בטבע על החלקים היה הכפל המוסיף חלק קודם על הכפל המוסיף חלקים | ||
| + | |- | ||
| + | |Therefore, it is necessary to begin with the discussion of the multiple superparticular ratio. | ||
| + | |style="text-align:right;"|ולזה יחוייב להקדים המאמר על הכפל המוסיף חלק | ||
| + | |- | ||
| + | |It is said that this ratio is conceived of two numbers, one of them is greater by once or many times the other and its part. | ||
| + | |style="text-align:right;"|ונאמר שזה היחס יראה משני מספרים אחד מהם יותר לאחר מדמיון אחד או דמיונים הרבה וכמו חלק ממנו | ||
| + | |- | ||
| + | | | ||
| + | :Such as: the five when they are related to the two, for the five are twice the two plus their half. <math>\scriptstyle{\color{blue}{5=\left(2\sdot2\right)+\left(\frac{1}{2}\sdot2\right)}}</math> | ||
| + | |style="text-align:right;"|כמו החמשה כאשר יוקשו אל שנים כי החמשה שני דמיוני שנים וכמו חציים | ||
| + | |- | ||
| + | |This species corresponds the two species of which it consists: | ||
| + | |style="text-align:right;"|וכבר יקח זה המין הדמוי משני המינים אשר הוא מורכב מהם | ||
| + | |- | ||
| + | | | ||
| + | *It corresponds to the multiple ratio: its multiplicity increases and the part remains the same. | ||
| + | |style="text-align:right;"|אמנם מה שיקח מיחס הכפל הנה ברבות כפלו והחלק אחד בלתי מומר | ||
| + | |- | ||
| + | | | ||
| + | :Such as: the double sesquialter ratio, the triple sesquialter ratio, the quadruple sesquialter ratio | ||
| + | |style="text-align:right;"|כמו {{#annot:double sesquialter|130|0eOa}}הכפל השניי המוסיף חצי{{#annotend:0eOa}} ו{{#annot:triple sesquialter|130|QhGF}}הכפל השלישיי המוסיף חצי{{#annotend:QhGF}} והכפל הרביעיי המוסיף חצי | ||
| + | |- | ||
| + | | | ||
| + | :and so on always, its multiples increase and the part remains the same. | ||
| + | |style="text-align:right;"|וכן תמיד ברבות הכפלים והחלק אחד לא יומר | ||
| + | |- | ||
| + | | | ||
| + | *It corresponds to the superparticular ratio: its parts are changed, but its multiples do not increase. | ||
| + | |style="text-align:right;"|ואמנם מה שיקחהו מדמיון המוסיף חלק יומרו חלקיו ולא יתרבו כפליו | ||
| + | |- | ||
| + | | | ||
| + | :Such as: the double sesquialter ratio, the double sesquitertian ratio, the double sesquiquartan ratio, the double sesquiquintan ratio | ||
| + | |style="text-align:right;"|כמו הכפל השניי המוסיף חצי ו{{#annot:double sesquitertian|130|eo7V}}הכפל השניי המוסיף שליש{{#annotend:eo7V}} ו{{#annot:double sesquiquartan|130|aP3C}}הכפל השניי המוסיף רביע{{#annotend:aP3C}} ו{{#annot:double sesquiquintan|130|KnWp}}הכפל השניי המוסיף חומש{{#annotend:KnWp}} | ||
| + | |- | ||
| + | | | ||
| + | :and so on, its parts are changed and its multiples do not increase. | ||
| + | |style="text-align:right;"|עוד כן יומרו חלקיו ולא יתרבו כפליו | ||
| + | |- | ||
| + | | | ||
| + | *It is composed from the sum of their two properties: when its multiples increase and its parts are changed. | ||
| + | |style="text-align:right;"|וכבר יתחדש לו והתרכבו מהם קבוץ שני סגולותיהם כאשר יתרבו כפליו ויומרו חלקיו | ||
| + | |- | ||
| + | | | ||
| + | :Such as: the double sesquialter ratio, the triple sesquitertian ratio, the quadruple sesquiquartan ratio | ||
| + | |style="text-align:right;"|כמו הכפל השניי המוסיף חצי ו{{#annot:triple sesquitertian|130|fzWx}}הכפל השלישי המוסיף שליש{{#annotend:fzWx}} והכפל הרביעיי המוסיף רביע | ||
| + | |- | ||
| + | | | ||
| + | :and so on, its multiples increase and its parts are changed. | ||
| + | |style="text-align:right;"|וכן תמיד יתרבו כפליו ויומרו חלקיו | ||
| + | |- | ||
| + | !Forming the species according to the superparticular ratio: | ||
| + | |style="text-align:right;"|ואמנם יתחדש המין האחד לדמוי מהמוסיף חלק לבד | ||
| + | |- | ||
| + | | | ||
| + | :*Arranging the natural numbers, beginning from 2, in a line. | ||
| + | |style="text-align:right;"|באשר תסדר המספרים הטבעיים אשר התחלתם ב' בטור | ||
| + | |- | ||
| + | | | ||
| + | :*Arranging the natural odd numbers, beginning from 5, in a line. | ||
| + | |style="text-align:right;"|עוד תסדר הנפרדים הטבעיים אשר התחלתם ה' בטור | ||
| + | |- | ||
| + | | | ||
| + | :*Then, each term is related to its corresponding, as seen in the example of the following diagram: | ||
| + | |style="text-align:right;"|ונקיש כל מדרגה אל הדומה לה כמו שתראה בזה הדמיון בזאת הצורה צורת הדמיון שנזכרנו | ||
| + | |- | ||
| + | |} | ||
| + | |||
| + | {|class="wikitable" style="margin-left: auto; margin-right: auto; text-align:center;" | ||
| + | |- | ||
| + | |כז||כו||כה||כד||כג||כב||כא||כ||יט||יח||יז||יו||טו||יד||יג||יב||יא||י||ט||ח||ז||ו||ה||ד||ג||ב | ||
| + | |- | ||
| + | |נה||נג||נא||מט||מז||מה||מג||מא||לט||לז||לה||לג||לא||כט||כז||כה||כג||כא||יט||יז||טו||יג||יא||ט||ז||ה | ||
| + | |} | ||
| − | + | {|class="wikitable" style="color:blue; margin-left: auto; margin-right: auto; text-align:center; font-size:92%;" | |
|- | |- | ||
| + | |27||26||25||24||23||22||21||20||19||18||17||16||15||14||13||12||11||10||9||8||7||6||5||4||3||2 | ||
| + | |- | ||
| + | |55||53||51||49||47||45||43||41||39||37||35||33||31||29||27||25||23||21||19||17||15||13||11||9||7||5 | ||
| + | |} | ||
| + | |||
| + | {| | ||
| + | |- | ||
| + | !Finding the species according to the multiple ratio: | ||
| + | |style="text-align:right;"|ותמצא המין האחד לדמוי מהכפל לבד | ||
| + | |- | ||
| + | | | ||
| + | :*Arranging a row beginning with the two numbers that produce the multiple superparticular ratio, which are 2 and 5 beneath it. | ||
| + | |style="text-align:right;"|כשתסדר טור באורך יהיה ראשיתו שני המספרים הפועלים ביחס הכפל המוסיף חלק והם ב' ותחתיו ה‫' | ||
| + | |- | ||
| + | | | ||
| + | :*Arranging beneath the 5 the subsequent terms of the natural odds successively downwards, so that these terms begin the columns. | ||
| + | |style="text-align:right;"|עוד תסדר תחת הה' מה שימשך לו ממדרגות הנפרד הטבעי ימשכו קצתם לקצת יורדים ותשים אלו המדרגות יתחילו בטורים לוקחים ברחב | ||
| + | |- | ||
| + | | | ||
| + | ::The terms of each line increase by the number of its first term. | ||
| + | |style="text-align:right;"|יתוספו מדרגות כל טור מהם כמנין המדרגה הראשונה ממנו | ||
| + | |- | ||
| + | | | ||
| + | :*Then, each of the terms of this table is related to its corresponding term, which is its parallel in the first line. | ||
| + | |style="text-align:right;"|עוד תקיש כל אחת ממדרגות זה המרובע באשר נכח לו והוא מקבילו מהטור הראשון | ||
| + | |- | ||
| + | | | ||
| + | :One finds that the multiple ratio is set in it without the the part growing, as seen here: | ||
| + | |style="text-align:right;"|שאתה תמצא בזה כבר העמדת בו יחס הכפל מבלתי שיצמח החלק וזה כמו שתראה בכאן | ||
| + | |- | ||
| + | | | ||
| + | :{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | |20||18||16||14||12||10||8||6||4||2 | ||
| + | |- | ||
| + | |50||45||40||35||30||25||20||15||10||5 | ||
| + | |- | ||
| + | |70||63||56||49||43||35||28||21||14||7 | ||
| + | |- | ||
| + | |90||81||72||63||54||45||36||27||18||9 | ||
| + | |- | ||
| + | |110||99||88||77||66||55||44||33||22||11 | ||
| + | |- | ||
| + | |130||117||104||91||78||65||52||39||26||13 | ||
| + | |- | ||
| + | |150||135||120||105||90||75||60||45||30||15 | ||
| + | |- | ||
| + | |170||153||136||119||102||85||68||51||34||17 | ||
| + | |- | ||
| + | |190||171||152||133||114||95||76||57||38||19 | ||
| + | |- | ||
| + | |210||189||168||147||126||105||84||63||42||21 | ||
| + | |} | ||
| | | | ||
| − | |style="text-align: | + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" |
| + | |- | ||
| + | |כ||יח||יו||יד||יב||י||ח||ו||ד||ב | ||
| + | |- | ||
| + | |נ||מה||מ||לה||ל||כה||כ||טו||י||ה | ||
| + | |- | ||
| + | |ע||סג||נו||מט||מב||לה||כח||כא||יד||ז | ||
| + | |- | ||
| + | |צ||פא||עב||סג||נד||מה||לו||כז||יח||ט | ||
| + | |- | ||
| + | |קי||צט||פח||עז||סו||נה||מד||לג||כב||יא | ||
| + | |- | ||
| + | |קל||קיז||קד||צא||עח||סה||נב||לט||כו||יג | ||
| + | |- | ||
| + | |קנ||קלה||קכ||קה||צ||עה||ס||מה||ל||טו | ||
| + | |- | ||
| + | |קע||קנג||קלו||קיט||קב||פה||סח||נא||לד||יז | ||
| + | |- | ||
| + | |קצ||קעא||קנב||קלג||קיד||צה||עו||נז||לח||יט | ||
| + | |- | ||
| + | |רי||קפט||קסח||קמז||קכו||קה||פד||סג||מב||כא | ||
| + | |} | ||
| + | |- | ||
| + | !Forming the species according to the two principles of which it consists | ||
| + | |style="text-align:right;"|ויתחדש זה המין האחד ליחס משני פנותיו אשר הוא מורכב מהם | ||
| + | |- | ||
| + | |That [species] whose multiples increase and whose parts are changed. | ||
| + | |style="text-align:right;"|והוא אשר יתרבו כפליו ויומרו חלקיו | ||
|- | |- | ||
| | | | ||
| − | ==== | + | :*Arranging the natural odd numbers, beginning from 5, in a red line. |
| + | |style="text-align:right;"|באשר תסדר הנפרדים הטבעיים אשר התחלתם מה' בטור הנרשם באודם | ||
| + | |- | ||
| + | | | ||
| + | ::We do not relate to it, but it is set as an inscription from which an example is taken. | ||
| + | |style="text-align:right;"|ולא נרצה להקיש בו אבל לשומו רושם לקחת דמיון ממנו | ||
| + | |- | ||
| + | | | ||
| + | :*Arranging a line: | ||
| + | |style="text-align:right;"|וזה שאנו נסדר טור אחד | ||
| + | |- | ||
| + | | | ||
| + | ::*The beginning of its terms is also 5. | ||
| + | |style="text-align:right;"|יהיה ראש מדרגותיו גם כן ה‫' | ||
| + | |- | ||
| + | | | ||
| + | ::*The second term is as its prdecessor, i.e. as 5, to which what is above it is added, i.e. from the preceding initial red line. | ||
| + | |style="text-align:right;"|והמדרגה השנית כמו אשר לפניה ר"ל כמו ה' מוסף עליו מה שלמעלה ממנו ר"ל מהטור הראשון האדום אשר לפניה | ||
| + | |- | ||
| + | | | ||
| + | ::*The third term as its prdecessor, i.e. as 10, to which what is above it is added, i.e. the second term of the red line. | ||
| + | |style="text-align:right;"|והמדרגה השלישית כמו אשר לפניה ר"ל כמו י' מוסף עליו מה שהוא למעלה ממנו ר"ל המדרגה השנית מהטור האדום | ||
| + | |- | ||
| + | | | ||
| + | ::*Proceeding likewise, until the end of the numbers that were set in the red line. | ||
| + | |style="text-align:right;"|וכן תעשה עד שיכלה אל סוף מה שהנחת מהמספרים בטור האדום | ||
| + | |- | ||
| + | | | ||
| + | :*As this second line is produced, so a line of the natural numbers beginning from 2 is set parallel to it. | ||
| + | |style="text-align:right;"|וכאשר הולדנו זה הטור השני כן סדרנו נכחו טור המספרים הטבעיים והתחלתו מב‫' | ||
| + | |- | ||
| + | | | ||
| + | :*Then, its terms are related to the terms of the line that was produced, leaving aside the red line. | ||
| + | |style="text-align:right;"|עוד נקיש בין מדרגותיו ובין מדרגות הטור אשר הולדנו ונעזוב הטור האדום | ||
| + | |- | ||
| + | |By relating them it is apparent how the species that corresponds to its two sides is produced - this is the third species of the multiple superparticular ratios. Examine it in the diagram we made and it will lead you straight: | ||
| + | |style="text-align:right;"|ויראה בהקשתם איך יתילד המין הלוקח הדמוי משני פאותיו וזה המין השלישי ממיני הכפל המוסיף חלק ותבחנהו בצורה שעשינו והתישר בו | ||
| + | |} | ||
| + | |||
| + | {|class="wikitable" style="margin-left: auto; margin-right: auto; text-align:center; font-size:70%;" | ||
| + | |- | ||
| + | |נט||נז||נה||נג||נא||מט||מז||מה||מג||מא||לט||לז||לה||לג||לא||כט||כז||כה||כג||כא||יט||יז||טו||יג||יא||ט||ז||ה|| | ||
| + | |- | ||
| + | |תתקא||תתמב||תשפה||תשל||תרעז||תרכו||תקעז||תקל||תפה||תמב||תא||שסב||שכה||רצ||רנז||רכו||קצז||קע||קמה||קכב||קא||פב||סה||נ||לז||כו||יז||י||ה | ||
| + | |- | ||
| + | |ל||כט||כח||כז||כו||כה||כד||כג||כב||כא||כ||יט||יח||יז||יו||טו||יד||יג||יב||יא||י||ט||ח||ז||ו||ה||ד||ג|| | ||
| + | |} | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: auto; text-align:center; color:blue; font-size:68%;" | ||
| + | |- | ||
| + | |59||57||55||53||51||49||47||45||43||41||39||37||35||33||31||29||27||25||23||21||19||17||15||13||11||9||7||5|| | ||
| + | |- | ||
| + | |901||842||785||730||677||626||577||530||485||442||401||362||325||290||257||226||197||170||145||122||101||82||65||50||37||26||17||10||5 | ||
| + | |- | ||
| + | |30||29||28||27||26||25||24||23||22||21||20||19||18||17||16||15||14||13||12||11||10||9||8||7||6||5||4||3|| | ||
| + | |} | ||
| + | |||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ===== The Discussion on the Fifth Ratio which is the Multiple Superpartient Ratio ===== | ||
| + | |||
| + | |style="text-align:right;"|<big>הדבור ביחס החמישי והוא יחס הכפלים המוסיף חלקים</big> | ||
| + | |- | ||
| + | |As was said, it is composed of the multiple ratio and the superpartient ratio. | ||
| + | |style="text-align:right;"|הוא כמו שאמרנו מורכב מיחס הכפל ומיחס המוסיף חלקים | ||
| + | |- | ||
| + | |Therefore, the technique of the production of this ratio is divided into three parts: | ||
| + | |style="text-align:right;"|ולזה גם כן כבר תחלק המלאכה בהולדת זה היחס לשלשה חלקים | ||
| + | |- | ||
| + | | | ||
| + | *Striving to reveal the quality of the multiple ratio by increasing its multiples, without changing the added parts. | ||
| + | |style="text-align:right;"|אם שתשתדל בם להראות סגולת הכפל ברבות כפליו מבלתי השתנות החלקים הנוספים או תמורתם | ||
| + | |- | ||
| + | | | ||
| + | *Teaching to reveal the quality of the superpartient ratio by changing the parts, without increasing the multiples. | ||
| + | |style="text-align:right;"|ואם שתתחכם בם להראות סגולת המוסיף חלקים בהמרת החלקים בלתי רבות הכפלים | ||
| + | |- | ||
| + | | | ||
| + | *Revealing both qualities in a continuous evident ratio as it is by nature. | ||
| + | |style="text-align:right;"|ואם שיראו בם שתי הסגולות יחס הראות נמשך כמו שהוא בטבע | ||
| + | |- | ||
| + | |The species whose multiples increase while its parts do not change is created when placing the two initial numbers that produce whichever multiple superpartient ratio one wishes: | ||
| + | |style="text-align:right;"|הנה המין אשר יצמחו כפליו ולא ישתנו חלקיו יתחדש כשנניח שני המספרים הראשונים הפועלים ליחס אי זה הכפל המוסיף חלקים שנרצה | ||
| + | |} | ||
| + | |||
| + | {|class="wikitable" style="margin-left: auto; margin-right: auto; text-align:center;" | ||
| + | |- | ||
| + | |לז||לה||לג||לא||כט||כז||כה||כג||כא||יט||יז||טו||יג||יא||ט||ז||ה|| | ||
| + | |- | ||
| + | |שסב||שכה||רצ||רנז||רכו||קצז||קע||קמה||קכב||קא||פב||סה||נ||לז||כו||יז||י||ה | ||
| + | |- | ||
| + | |יט||יח||יז||יו||טו||יד||יג||יב||יא||י||ט||ח||ז||ו||ה||ד||ג||ב | ||
| + | |} | ||
| + | |||
| + | {|class="wikitable" style="color:blue; margin-left: auto; margin-right: auto; text-align:center;" | ||
| + | |- | ||
| + | |37||35||33||31||29||27||25||23||21||19||17||15||13||11||9||7||5|| | ||
| + | |- | ||
| + | |362||325||290||257||226||197||170||145||122||101||82||65||50||37||26||17||10||5 | ||
| + | |- | ||
| + | |19||18||17||16||15||14||13||12||11||10||9||8||7||6||5||4||3||2 | ||
| + | |} | ||
| + | |||
| + | {| | ||
| + | |- | ||
| + | |For example: the multiple superbitertian ratio | ||
| + | |style="text-align:right;"|וכאלו נרצה יחס הכפל המוסיף שני שלישים | ||
| + | |- | ||
| + | | | ||
| + | :*Placing the two initial numbers that produce them, which are 3 and 8. | ||
| + | |style="text-align:right;"|הנה נניח שני המספרים הראשונים הפועלים להם והם ג' וח‫' | ||
| + | |- | ||
| + | | | ||
| + | :*For the rest of the multiple superbitertian ratios: leaving the smaller, and arranging a row beneath the 8 whose terms begin from 8 and increase by the smaller number, i.e. increse by threes. | ||
| + | |style="text-align:right;"|וכאשר נרצה שאר הכפלים המוסיפים שני שלישים נעזוב הקטן על ענינו ונסדר תחתיו טור באורך לוקח תחת ח' יתחילו מדרגותיו מח' יתוספו במניין אחרי המספר הקטן ר"ל שיתוספו ג' ג‫' | ||
| + | |- | ||
| + | | | ||
| + | :*Relating each of them to the first term, i.e. to the first number, which is 3, because they are related to it by the multiple superbitertian ratio. | ||
| + | |style="text-align:right;"|עוד נקיש כלם במדרגה הראשונה ר"ל במספר הקטן אשר הוא ג' כי הם יהיו אליו ביחס הכפל המוסיף אותם החלקים הראשונים | ||
| + | |- | ||
| + | | | ||
| + | :Thus the multiples are produced according to their natural succession, without the parts increasing or changing. | ||
| + | |style="text-align:right;"|וכבר התילדו הכפלים כפי המשכם בטבע מבלתי שיתוספו החלקים או יומרו | ||
| + | |- | ||
| + | |By this technique whichever multiple superpartient ratio one wishes is produced. | ||
| + | |style="text-align:right;"|ובזאת המלאכה יתילדו איזה מן הכפלים המוסיף חלקים שתרצה | ||
| + | |- | ||
| + | |Such as: the multiple supertriquartan ratio, or the multiple supertriquintan ratio, or whichever one wishes of this. | ||
| + | |style="text-align:right;"|כמו הכפל המוסיף שלשה רביעיות או שלשה חמשיות או מה שתרצה מזה | ||
| + | |- | ||
| + | |You will be well guided in it in the example given here. | ||
| + | |style="text-align:right;"|ותתישר בו במשל אשר המשלתיו לך הנה | ||
| + | |- | ||
| + | |The production of the species whose parts change and multiply by a natural successive reproduction according to the increase of number from which their names are derived [= the denominator], while the number of its multiples does not change: | ||
| + | |style="text-align:right;"|ואמנם איך יתילד המין אשר יומרו חלקיו ויעתקו ברבות המספר אשר ממנו יגזרו שמותיהם העתק נמשך בטבע מבלתי שיומר מנין כפליו | ||
| + | |- | ||
| + | |Its technique is divided also into two parts | ||
| + | |style="text-align:right;"|זה גם כן תחלק מלאכתו לשני חלקים | ||
| + | |- | ||
| + | | | ||
| + | :*Following the production of the first species of the superpartient ratio, as their natural succession. | ||
| + | |style="text-align:right;"|לפי שאנו אם שנלך בזה אל תולדת המינים הראשונים מהמוסיף חלקים כפי המשכם בטבע | ||
| + | |- | ||
| + | | | ||
| + | ::Such as: the superbipartient ratio, the supertripartient ratio, the superquadripartient ratio, and what follows. | ||
| + | |style="text-align:right;"|כמו המוסיף שני חלקים עוד {{#annot:supertripartient|128|EHqW}}המוסיף שלשה חלקים{{#annotend:EHqW}} עוד {{#annot:superquadripartient|128|GDCW}}המוסיף ארבעה חלקים{{#annotend:GDCW}} עוד מה שילווה לזה | ||
| + | |- | ||
| + | | | ||
| + | :*Following the production of one species among them, by extracting each ratio that is denoted by the name of that same ratio. | ||
| + | |style="text-align:right;"|ואם שנלך אל הולדת מין אחת מהם בהוצאת כל יחס יפל תחת שם אותו המין | ||
| + | |- | ||
| | | | ||
| + | ::Such as: the superbipartient ratio alone, or the supertripartient ratio alone. | ||
| + | |style="text-align:right;"|כמו המוסיף שני חלקים לבד והמוסיף שלשה חלקים לבד | ||
| + | |- | ||
| + | |The technique that leads to the first species of the superpartient ratio according to their natural succession, without increasing the multiples: | ||
| + | |style="text-align:right;"|אבל המלאכה אשר תביא אל המינים הראשונים מהמוסיף חלקים כפי המשכם בטבע מבלתי שירבו הכפלים | ||
|- | |- | ||
| | | | ||
| − | ===== | + | :*Arranging the natural numbers in a line, beginning from 3. |
| + | |style="text-align:right;"|אמנם היא כשנסדר המספרים הטבעיים שהתחלתם ג' בטור | ||
| + | |- | ||
| + | | | ||
| + | :*Placing beneath 3 the number that produces with it the initial superpartient ratio. | ||
| + | |style="text-align:right;"|ותניח תחת ג' המספר אשר יפעל עמם תחלת יחס המוסיף חלקים | ||
| + | |- | ||
| + | | | ||
| + | :*Completing the greater line with terms added by threes. | ||
| + | |style="text-align:right;"|עוד נשלים הטור הגדול במדרגות יתוספו ג' ג‫' | ||
| + | |- | ||
| + | | | ||
| + | ::*Once - if we want the double ratio. | ||
| + | |style="text-align:right;"|פעם א' אם רצינו שיהיה הכפל שניי | ||
| + | |- | ||
| + | | | ||
| + | ::*twice - if we want the triple ratio. | ||
| + | |style="text-align:right;"|ואם רצינו אותו שלישיי כזה שני פעמים | ||
| + | |- | ||
| + | | | ||
| + | ::*thrice - if we want the quadruple ratio | ||
| + | |style="text-align:right;"|ואם רצינו אותו רביעיי שלשה פעמים | ||
| + | |- | ||
| + | | | ||
| + | ::*So on for what follows. | ||
| + | |style="text-align:right;"|וכן מה שאחר זה | ||
| + | |- | ||
| + | | | ||
| + | :*Relating each term to what is above it, i.e. its counterpart in the first line | ||
| + | |style="text-align:right;"|עוד נקיש כל מדרגה באשר למעלה ממנה ר"ל הדומה לה מהטור הראשון | ||
| + | |- | ||
| + | |Thus, the multiple superpartient ratio is revealed according to the natural production of the first species of the superpartient ratio, without increasing the multiples. | ||
| + | |style="text-align:right;"|הנה יראה לנו יחס הכפל המוסיף חלקים כפי התילד המינים הראשונים מהמוסיף חלק בטבע בלתי שיתרבו הכפלים | ||
| + | |- | ||
| + | |As seen in this example - examine it and you will find it with God's help. | ||
| + | |style="text-align:right;"|וזה כפי מה שתראה בזה המשל ותבחנהו ותמצאהו בע"ה | ||
| + | |} | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: auto; text-align:center;" | ||
| + | |- | ||
| + | | ||יח||יז||יו||טו||יד||יג||יב||יא||י||ט||ח||ז||ו||ה||ד|| | ||
| + | |- | ||
| + | |נג||נ||מז||מד||מא||לח||לה||לב||כט||כו||כג||כ||יז||יד||יא||ח||ג | ||
| + | |} | ||
| + | {|class="wikitable" style="color:blue; margin-left: auto; margin-right: auto; text-align:center;" | ||
| + | |- | ||
| + | | ||18||17||16||15||14||13||12||11||10||9||8||7||6||5||4|| | ||
| + | |- | ||
| + | |53||50||47||44||41||38||35||32||29||26||23||20||17||14||11||8||3 | ||
| + | |} | ||
| − | + | {| | |
| + | |- | ||
| + | |The production of the species of the multiple superbipartient for example, or the species of the multiple supertripartient, or the species of the multiple superquadripartient, or others, without increasing the multiples and without changing the number of the parts, but changing the number from which they are derived according to natural growth: | ||
| + | |style="text-align:right;"|ואמנם איך יתילדו מיני הכפלים המוסיפים שני חלקים דרך משל או מיני הכפל המוסיף שלשה חלקים או מיני המוסיף ארבעת חלקים או זולת אלו מבלתי שיתרבו הכפלים ולא ישתנה מנין החלקים אבל ישתנה בצמיחה הטבעית המנין אשר הם נגזרים ממנו | ||
| + | |- | ||
| + | | | ||
| + | :*Extracting those having the same multiples beneath the superpartient ratio of the same parts, as shown in the chapter on the superpartient ratio. | ||
| + | |style="text-align:right;"|וזה כשנוציא המתדמים בכפלם תחת המוסיף אותם החלקים כמו שכבר גלינו בשער המוסיף חלקים | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::*the smaller number in each term is set above it greater counterpart - if you want the double ratio. |
| + | |style="text-align:right;"|עוד ינשא המספר הקטן מכל מדרגה על הגדול אשר נכחו אם תרצה שיהיה הכפל השניי | ||
|- | |- | ||
| | | | ||
| − | ===== | + | ::*double the smaller - if you want the triple ratio. |
| + | |style="text-align:right;"|ואם תרצה שיהיה שלישיי תשא כפל הקטן | ||
| + | |- | ||
| + | | | ||
| + | ::*thrice the smaller number is set above - if you want the quadruple ratio. | ||
| + | |style="text-align:right;"|ואם תרצה אותו רבעיי תשא שלשה כפלי הקטן | ||
| + | |- | ||
| + | | | ||
| + | ::*And so on for all that follows in all the terms of the greater line. | ||
| + | |style="text-align:right;"|וכן מה שאחר זה בכל מדרגות הטור הגדול | ||
| + | |- | ||
| + | | | ||
| + | :*Relating each term to its counterpart. | ||
| + | |style="text-align:right;"|עוד נקיש כל מדרגה בנכחית לה | ||
| + | |- | ||
| + | |The superpartient ratio of these parts is found without increasing the multiples or multiplying the desired number. | ||
| + | |style="text-align:right;"|שאתה תמצא המוסיף אותם החלקים בלתי שיוסיפו הכפלים או יעתקו מהמנין אשר תרצה | ||
| + | |- | ||
| + | |Examine what was mentioned in both descriptions in the chaper on the superpartient ratio, which are the species of the superpartient ratio whose number does not change, and you will be well guided by them in the production of this species of the multiple superpartient ratio by observing carefully what was noted. | ||
| + | |style="text-align:right;"|ובחן מה שזכרתיו לך בשני הרשמים לפניך בשער המוסיף חלקים והם טור מיני המוסיף חלקים לא יומר מנינם ותתישר מהם להולדת זה המין מהכפל המוסיף חלקים כאשר תטיב להתבונן במה שזכרנוהו | ||
| + | |- | ||
| + | |The third type of the first division mentioned in the beginning of this chapter - the production of the species whose multiples increase and whose parts change: | ||
| + | |style="text-align:right;"|ואמנם המין השלישי מהחלוקה הראשונה הנזכרת בפתיחת זה הספר והוא איך יתילדו המינים אשר יצמחו כפליהם וישתנו חלקיהם | ||
| + | |- | ||
| + | |Since the change of the parts is either by the constancy of their number and the natural increase of their values, or by the increase of their number and the increase of their values together, the technique of extracting them is necessarily divided into two parts: | ||
| + | |style="text-align:right;"|הנה לפי שהשתנות החלקים אם שיהיה בקיום מנינם והתרבות הגעותיהם התרבות טבעי ואם שיהיה בהתרבות מנינם והתרבות הגעותיהם יחד יחוייב שתחלק המלאכה בהוצאת זה לשני חלקים | ||
| + | |- | ||
| + | | | ||
| + | *The production of the species in which the number of the parts is constant and their values increase naturally: | ||
| + | |style="text-align:right;"|אבל יתילד המין אשר יתקיים בו מניין החלקים וישתנו הגעותיהם בהתרבות הטבעיי | ||
| + | |- | ||
| + | | | ||
| + | :Its technique is by extracting two lines of the superpartient ratio whose number, i.e. the number of the added parts, is as the number you wish to ascribe. | ||
| + | |style="text-align:right;"|תהיה מלאכתו כאשר נוציא שני טורים מיחס המוסיף חלקים מנינם ר"ל מנין החלקים הנוספים כמניין אשר תרצה לחייבו | ||
| + | |- | ||
| + | | | ||
| + | :You will be well guided by it in the two lines written in this manner in the chapter on the superpartient ratio, which are the two lines in which commensurability and incommensurability are noted. | ||
| + | |style="text-align:right;"|ותתישר בזה בשני הטורים הנרשמים בדומה זה בשער המוסיף חלקים והם שני הטורים אשר הוכר בם השתוף מהבלתי שתוף | ||
| + | |- | ||
| + | | | ||
| + | :Then, above each of the terms of the greater numbers, set the product of its counterpart among the terms of the smaller numbers by the number of the values of the parts less one. | ||
| + | |style="text-align:right;"|עוד ינשא על כל מדרגה מן המספרים הגדולים מה שבדומה לה מן המדרגות המספרים הקטנים מוכה במנין הגעות החלקים אלא אחד | ||
| + | |- | ||
| + | | | ||
| + | :When this is done for all the terms of the greater line - each term of which is related to its counterpart among the terms of the smaller line, i.e. whose numbers are the smaller - one finds all this in the multiple superpartient ratio, while the values of their parts are the numbers of the multiples, i.e. the number of the parts does not change. | ||
| + | |style="text-align:right;"|וכאשר עשית זה בכל מדרגות הטור הגדול הקשת בין כל מדרגה ממנו ודומה לה ממדרגות הטור הקטן ר"ל בעל המספרים הקטנים שאתה תמצא כל זה ביחס הכפל המוסיף חלקים הגעתם הוא מנין הכפלים ר"ל שמנין החלקים לא יומרו | ||
| + | |- | ||
| + | | | ||
| + | *The second part of this type - in which the multiples, the number of the parts and their values increase together. | ||
| + | |style="text-align:right;"|ואמנם החלק השני מזה המין והוא אשר יתרבו בו הכפלים ומנין החלקים והגעתם יחד | ||
| + | |- | ||
| + | | | ||
| + | :Their production technique: | ||
| + | :*Placing the natural numbers, beginning from three, in a line. | ||
| + | |style="text-align:right;"|הנה מלאכת הולדתם בהנחת המספרים הטבעיים אשר התחלתם ג' בטור | ||
| + | |- | ||
| + | | | ||
| + | :*Placing 8 beneath the 3 and completing the second line, whose beginning is 8, by adding the natural odd numbers, beginning from 7: | ||
| + | |style="text-align:right;"|עוד תניח תחת ג' ח' ותשלים הטור השני אשר התחלתו ח' בתוספת הנפרדים הטבעיים אשר התחלתם ז‫' | ||
| + | |- | ||
| + | | | ||
| + | ::*secone term: <math>\scriptstyle{\color{blue}{7+8=15}}</math> | ||
| + | |style="text-align:right;"|ותוסיף ז' על ח' ותעמיד מה שהתקבץ במדרגה השנית וזה ט"ו | ||
| + | |- | ||
| + | | | ||
| + | ::*[third] term: <math>\scriptstyle{\color{blue}{15+9=24}}</math> | ||
| + | |style="text-align:right;"|עוד תוסיף על ט"ו ט' ותעמיד מה שהתקבץ במדרגה | ||
| + | |- | ||
| + | | | ||
| + | ::*fourth term: third term + 11 | ||
| + | |style="text-align:right;"|וכן תוסיף על השלישית י"א ותעמיד מה שהתקבץ ברביעית | ||
| + | |- | ||
| + | | | ||
| + | :*Proceeding like this in the rest of the terms of the second line, until reaching the counterpart of the end of the natural numbers placed in the first line. | ||
| + | |style="text-align:right;"|ותעשה כמו זה בשאר מדרגות הטור השני עד שתגיע לנכח סוף מה שהנחת מן המספרים הטבעיים בטור הראשון | ||
| + | |- | ||
| + | | | ||
| + | :*Relating each term to its counterpart, as seen in these two lines: | ||
| + | |style="text-align:right;"|עוד נקיש כל מדרגה בדומה לה כמו שתראה בשני אלו הטורים | ||
| + | |} | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: auto; text-align:center;" | ||
| + | |- | ||
| + | |טו||יד||יג||יב||יא||י||ט||ח||ז||ו||ה||ד||ג | ||
| + | |- | ||
| + | |רכד||קצה||קסח||קמג||קכ||צט||פ||סג||מח||לה||כד||טו||ח | ||
| + | |} | ||
| + | {|class="wikitable" style="color:blue; margin-left: auto; margin-right: auto; text-align:center;" | ||
| + | |- | ||
| + | |15||14||13||12||11||10||9||8||7||6||5||4||3 | ||
| + | |- | ||
| + | |224||195||168||143||120||99||80||63||48||35||24||15||8 | ||
| + | |} | ||
| − | + | {| | |
| + | |- | ||
| + | |The species of the multiple superpartient ratio, of which the multiples, the number of the parts and their values all increase naturally, are revealed with God's help. | ||
| + | |style="text-align:right;"|ויראו לך בע"ה מיני הכפל המוסיף חלקים אשר התרבו כפליהם ומנין חלקיהם והגעות חלקיהם כמו כן התרבות טבעי נמשך | ||
| + | |- | ||
| + | |Thus, the five ratios and the ten numeral relations were discussed and stated in a remarkable discussion, as needed, without multiplicity and lengthiness. | ||
| + | |style="text-align:right;"|הנה כבר דברנו על היחסים החמש וההקשות המספריות העשרה ואמרנו בם מאמר מופלג כפי הצורך מבלתי רבוי ואריכות | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== The Discussion on the Technique of Producing the Ratios from Equality ==== | ||
| + | |||
| + | |style="text-align:right;"|<big>המאמר על התחבולה בהולדת היחסים מן השווי</big> | ||
| + | |- | ||
| + | |Following what was said we present the way to produce these five ratios from equality by a basic valid coherent technique, which follows natural matters in its generality and truth of interpretation. | ||
| + | |style="text-align:right;"|וכבר ימשך למה שקדם מדברינו שנביא הצד בהולדת אלו היחסים החמש מן השווי בתחבולה שרשית קיימת בלתי מבולבלת תדמה הענינים הטבעיים בכללותה ואמתת פירושה | ||
| + | |- | ||
| + | |The fruit of what will be noted of this is very precious and plentiful. | ||
| + | |style="text-align:right;"|והפרי במה שנזכור מזה יקר ונכבד מאד | ||
| + | |- | ||
| + | |All the more so for whoever examines my investigation of the metaphor of the knowledge of good. | ||
| + | |style="text-align:right;"|וכל שכן אצל מי שבחן מה שאעיין בו מדמיון הידיעה לטוב | ||
| + | |- | ||
| + | |For the good is determinate in the soul, its likeness and relation are known, and the souls are drawn to it. | ||
| + | |style="text-align:right;"|כי הטוב אצל הנפש מוגבל וידוע המשלו וחברו ונמשכו לו הנפשות | ||
| + | |- | ||
| + | |Know, that it is first for all that is uncompounded by itself, preserving its perception in all that its nature generates, and that the evil, in its disgrace, is infinite, indeterminate, does not branch out from a root, and does not follow an interpretation, indeed, it is a change of the nature, which is good. | ||
| + | |style="text-align:right;"|ותדע שהוא ראשון לכל מה שיתפרד מעצמו מתמיד ההרגש בכל מה שיתילד מטבעו ושהרע בגנותו בלתי בעל תכלית ולא מגיע אל גבול ולא יסתעף משרש ולא נמשך על פירוש אמנם הוא שנוי הטבע אשר הוא טוב | ||
| + | |- | ||
| + | |Their deviation from their primary nature is a changing deviation, indeterminate, and without order. | ||
| + | |style="text-align:right;"|הנה היציאה מטבעם הראשון להם יציאה מתחלפת לא תכלל בגבול ולא תתקיים בסדר | ||
| + | |- | ||
| + | |Therefore, a rational capacity does not hold them by a rational hold, but recognizes them when they deviate from nature. | ||
| + | |style="text-align:right;"|ולזה לא יאחזהו כח מדבר אחיזה מושכלת אבל יכירהו בהתחלפו לטבע | ||
| + | |- | ||
| + | |For example: the rust in the transparent mirror and the injustice of the right soul that are incomprehensible by way of definition and determination, since they have no essence, but are known by the change of the substance in which they were set and embedded. | ||
| + | |style="text-align:right;"|דרך משל אומר כחלודה במראה הספירית והעול בנפש הישרה שהם בלתי מושגים בדרך הגדר וההגבלה אחר שאין עצמיות להם ואמנם יודעו בהשתנות העצם אשר נקבעו ונטבעו בו | ||
| + | |- | ||
| + | |Yet, if we find determined ways for these five ratios, by which they are produced from equality and, inversely, reduced to equality, whereas equality is undoubtedly good and virtue, then the ratios also share the same virtue and joined to the same good. | ||
| + | |style="text-align:right;"|אבל אם אנחנו מצאנו לאלו היחסים החמש דרכים מוגבלים בהם יתילדו מהשווי ויותכו בהפוכם אל השווי והיה השווי בלי ספק טוב וחשיבות היו היחסים גם כן משותפים לאותו החשיבות ונקשרים באותו הטוב | ||
| + | |- | ||
| + | |Since their production from it is ordered, follows a determination, and preserves the capacity of their root, from which they are produced, as the seed preserves the capacity of the tree, since the seed becomes a tree also. | ||
| + | |style="text-align:right;"|אחר שהיה התילדם ממנו מסודר נמשך על גבול שומר לכח שרשם אשר ממנו יתילדו כמו שישמור הגרגיר כח האילן אחר שכבר ישוב גם כן הגרגיר אילן | ||
| + | |- | ||
| + | |So these ratios are produced from equality and return to equality, however this is not by accident, rather a technique and a correlation are needed, as the cultivation of land is needed for the seed and the tree to sprout. | ||
| + | |style="text-align:right;"|וכן אלו היחסים יתילדו מן השווי וישובו כמו כן אל השווי אבל לא יהיה זה בהזדמן אבל כבר נצטרך בו אל מלאכה וחבור כמו שנצטרך בענין הזרע והאילן על עבודת הקרקע קודם שיצא | ||
| + | |- | ||
| + | |For it is impossible to reach the end of an assumption, be it a theoretical assumption or a practical assumption, except by using the assistance of external things, which have some similarity to that assumption, and are related in some determinate order, through the guidance of which we reach the assumption. | ||
| + | |style="text-align:right;"|כי אי אפשר להגיע אל תכלית דרוש מן הדרושים דרוש מדעי היה או דרוש מעשי אם לא בהעזר מדברים מחוץ יהיה להם קצת התדמות לאותו הדרוש ויחוברו על סדר מה מוגבל נגיע עם ההדרכה עליהם אל הדרוש | ||
| + | |- | ||
| + | |Because of this, Euclid stated the relation as one of the things from which knowledge exists and by which practice is reached. | ||
| + | |style="text-align:right;"|ולזה שם אקלידס החבור אחד מהדברים אשר מהם תהיה הידיעה ובהם נגיע אל המעשה | ||
| + | |- | ||
| + | |Therefore, in the production of each of these ratios, we also place three numbers in three terms, in order to reach the relation through them. | ||
| + | |style="text-align:right;"|ולזה גם כן נניח אנחנו בהולדת כל אחד מאלו היחסים שלשה מספרים בשלשה מדרגות להגיע בם אל ההתיחסות | ||
| + | |- | ||
| + | |For the least relation possible is in three determinations. | ||
| + | |style="text-align:right;"|כי ההתיחס בפחות מה שיהיה הוא בשלשה גבולים | ||
| + | |- | ||
| + | |In relation, one is assisted by things that are apart from that which has the similarity of the ratio. | ||
| + | |style="text-align:right;"|ואמנם יעזרו בהתיחסות מהדברים אשר מחוץ למה שבו מהתדמות היחס | ||
| + | |- | ||
| + | |Since it was already said that the things that assist us are apart from the investigation of the assumption, they should be similar to the assumption. | ||
| + | |style="text-align:right;"|ולפי שאנו כבר אמרנו שהדברים אשר נעזר בם מחוץ אל החקירה מהדרוש ראוי שיהיו מתדמים לדרוש | ||
| + | |- | ||
| + | |These three terms are called first, second and third. | ||
| + | |style="text-align:right;"|ויקראו אלו שלשת המדרגות ראשונה ושניה ושלישית | ||
| + | |- | ||
| + | |Each term among them is limited by a determination which it does not surpass: | ||
| + | |style="text-align:right;"|ותדבק כל מדרגה מהן בגבול לא תעברהו | ||
| + | |- | ||
| + | | | ||
| + | *The determination of the first term is that one should always place beneath it the same as the number in it. [<math>\scriptstyle{\color{blue}{a_1}}</math>] | ||
| + | |style="text-align:right;"|אם הגבול במדרגה הראשונה הוא שנניח תחתיה לעולם כמו המספר אשר בה | ||
| + | |- | ||
| + | | | ||
| + | *The determination of the second term is that one should always place beneath it the same as the number in it plus the same as the number in the first term. [<math>\scriptstyle{\color{blue}{a_2+a_1}}</math>] | ||
| + | |style="text-align:right;"|ואמנם הגבול במדרגה השנית הוא שנניח תחתיה לעולם כמו המספר אשר בה וכמו המספר אשר במדרגה הראשונה | ||
| + | |- | ||
| + | | | ||
| + | *The determination of the third term is that one should always place beneath it the same as the number in it plus the same as the number in the first term and double that which is in the second term. [<math>\scriptstyle{\color{blue}{a_3+a_1+2\left(a_2+a_1\right)}}</math>] | ||
| + | |style="text-align:right;"|ואמנם הגבול אשר במדרגה השלישית הוא שנניח תחתיה כמו המספר אשר בה וכמו המספר אשר במדרגה הראשונה וכפל אשר במדרגה השנית | ||
| + | |- | ||
| + | |This is the general technique by which each of these ratios is produced. | ||
| + | |style="text-align:right;"|זה הוא מה שהוא כולל במלאכה אשר בה יתילד כל אחד מאלו היחסים | ||
| + | |- | ||
| + | |Yet, what is particular in it for each of these ratios is: | ||
| + | |style="text-align:right;"|ואמנם מה שהוא מיוחד בה לכל אחד מאלו היחסים | ||
| + | |- | ||
| + | | | ||
| + | *The particular in this technique for the production of the multiple ratio is that the first given numbers in the three terms, from which this ratio is produced, are equal numbers, preserving equality in their relation. | ||
| + | |style="text-align:right;"|כי אשר תיחד המלאכה בהולדת יחס הכפל השווי שיהיו המספרים הראשונים המונחים במדרגות הג' אשר מהם יתילד זה היחס מספרים שוים שומרים השווי בהצטרפם | ||
| + | |- | ||
| + | | | ||
| + | :*When the aforementioned general condition is fulfilled, the numbers in the double ratio are revealed, and this is the first of the species of this ratio. | ||
| + | |style="text-align:right;"|וכשנשמור בם התנאי הכולל הנזכר יראו אלינו מספרים ביחס הכפל השניי והיה המין הראשון ממיני זה זה היחס | ||
| + | |- | ||
| + | | | ||
| + | :*If this resulting species is placed as given, and the aforementioned general condition is added, i.e. the formation of the three determinations in the three terms, then the second species of the multiple ratio, which is the triple ratio, is revealed. | ||
| + | |style="text-align:right;"|ואם שמנו זה המין הנראה מונח והוספנו בו התנאי הכולל הנזכר ר"ל העשות הגבולים השלשה במדרגות השלשה יראה לנו המין השני ממיני הכפל והוא הכפל השלישיי | ||
| + | |- | ||
| + | | | ||
| + | :*If it then placed as given, and the first procedure is repeated for it, the third species, which is the quadruple ratio, is revealed. | ||
| + | |style="text-align:right;"|ואם שמנוהו גם כן מונח והשבנו בו במעשה הראשון יראה לנו המין השלישי והוא הכפל הרביעיי | ||
| + | |- | ||
| + | | | ||
| + | :Accordingly, we do not stray from this procedure, so the species of the multiple ratio are revealed in their natural succession. | ||
| + | |style="text-align:right;"|וכפי זה הדרך לא נסור מזה המעשה יראו לנו מיני הכפל ראשון ראשון כפי המשכם בטבע | ||
| + | |- | ||
| + | | | ||
| + | :Examine this in these two lines: | ||
| + | |style="text-align:right;"|ובחן זה בשני טורים אלו | ||
| + | |- | ||
| + | | | ||
| + | :{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | |1||1||1|| | ||
| + | |- | ||
| + | |4||2||1||first | ||
| + | |- | ||
| + | |9||3||1||second | ||
| + | |- | ||
| + | |16||4||1||third | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | |א||א||א|| | ||
| + | |- | ||
| + | |ד||ב||א||ראשון | ||
| + | |- | ||
| + | |ט||ג||א||שני | ||
| + | |- | ||
| + | |יו||ד||א||ג | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | :Take them as a basis for what follows them. | ||
| + | |style="text-align:right;"|וקחם לשרש למה שימשך להם | ||
| + | |- | ||
| + | | | ||
| + | *The particular in this technique for the production of the superparticular ratio is that the given numbers, from which one wishes to produce the superparticular ratio, are related in the multiple ratio, the greater is in the first place, and the smaller is in the third place, then one employs the aforementioned general repetition. | ||
| + | |style="text-align:right;"|ואמנם אשר הוא מיוחד בזאת המלאכה להוליד יחס המוסיף חלק הנה הוא שיהיו המספרים המונחים אשר מהם תרצה להוליד המוסיף חלק מתיחסים ביחס הכפל ויהיה הגדול מהם במקום הראשון והקטן מהם במקום השלישי עוד תעשה בם הסבוב הכולל אשר זכרנו | ||
| + | |- | ||
| + | | | ||
| + | :For, what follows from this is that the species of the superparticular ratio are seen according to their natural succession. | ||
| + | |style="text-align:right;"|כי ימשך לזה שיראו אלינו מיני המוסיף חלק כפי המשכם בטבע | ||
| + | |- | ||
| + | | | ||
| + | :*If the given numbers were of the first species of the multiple ratio, i.e. the double ratio, the [first] species of the superparticular ratio will be seen from them, i.e., the sesquialter ratio. | ||
| + | |style="text-align:right;"|ר"ל שהמספרים המונחים אם היו עליהם תחלת מיני הכפל ר"ל השניי יראו מהם מיני המוסיף חלק ר"ל המוסיף חצי | ||
| + | |- | ||
| + | | | ||
| + | :*If they were of the second species of the multiple ratio, i.e. the triple ratio, the second species of the superparticular ratio will be seen from them, i.e., the sesquitertian ratio. | ||
| + | |style="text-align:right;"|ואם היו על היחס השני ממיני הכפל ר"ל השלישיי יראה לנו גם כן השני מהמוסיפי חלק ר"ל המוסיף שליש | ||
| + | |- | ||
| + | | | ||
| + | :The same for what follows. | ||
| + | |style="text-align:right;"|וכמו כן מה שאחר זה | ||
| + | |- | ||
| + | |Examine it from this diagram: | ||
| + | |style="text-align:right;"|ותבחנהו מזאת הצורה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color: blue; text-align:center;" | ||
| + | |- | ||
| + | |1||2||4 | ||
| + | |- | ||
| + | |9||6||4 | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | |א||ב||ד | ||
| + | |- | ||
| + | |ט||ו||ד | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | *The particular for the production of the superpartient ratio is that the given numbers are related in the superparticular ratio, the greater in the first place and the smaller in the third place, then one employs the aforementioned general repetition. | ||
| + | |style="text-align:right;"|ואשר ייחד תולדת המוסיף חלקים הוא שיהיו המספרים המונחים מתיחסי' ביחס המוסיף חלק ויהיה הגדול מהם במקום הראשון והקטן מהם במקום השלישי עוד נעשה בם הסבוב הכולל הנזכר | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|הוא | + | :For it reveals the numbers in a species of the superpartient ratio, arranged approximately to the natural arrangement of the given superparticular ratio, as this: |
| + | |style="text-align:right;"|כי הוא יראה לנו המספרים על יחס מין מהמוסיף חלקים מסודרים בקירוב מהטבע בסדור המוסיף חלק המונח וזה יהיה כן | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color: blue; text-align:center;" | ||
| + | |- | ||
| + | |4||6||9 | ||
|- | |- | ||
| + | |25||15||9 | ||
| + | |} | ||
| | | | ||
| − | == | + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" |
| + | |- | ||
| + | |ד||ו||ט | ||
| + | |- | ||
| + | |כה||טו||ט | ||
| + | |} | ||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *The particular for the production of the multiple superparticular ratio is that the given numbers are related also in the superparticular ratio, but the greater is in the third place and the smaller is in the first place. |
| + | |style="text-align:right;"|ואשר ייחד הולדת יחס הכפל המוסיף חלק הוא שיהיו המספרים המונחים מתיחסים ביחס המוסיף חלק גם כן אלא שהגדול מהם יהיה במקום השלישי והקטן מהם במקום הראשון | ||
| + | |- | ||
| + | | | ||
| + | :The general conditional repetition reveals a species of the multiple superparticular ratio in approximation to nature, i.e. from the beginning of the species of the multiple superparticular ratio according to the terms of the given species of the superparticular ratio, and so it is: | ||
| + | |style="text-align:right;"|ויראה לנו הסבוב הכולל המותנה מין מהכפל המוסיף חלק הוא בקרוב מהטבע ר"ל מהתחלת מיני הכפל המוסיף חלק בכמו מדרגת המין המוסיף חלק המונח ויהי כן | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color: blue; text-align:center;" | ||
| + | |- | ||
| + | |9||6||4 | ||
| + | |- | ||
| + | |25||10||4 | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | |ט||ו||ד | ||
| + | |- | ||
| + | |כה||י||ד | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | *The particular for the production of the multiple superpartient ratio is that the given numbers are related in the superpartient ratio, the smaller in the first place and the greater in the third place. | ||
| + | |style="text-align:right;"|ואשר ייחד הולדת יחס הכפל המוסיף חלקים הוא שיהיו המספרים המונחים מתיחסים יחס המוסיף חלקים ויהיה הקטן מהם במקום הראשון והגדול מהם במקום השלישי | ||
| + | |- | ||
| + | | | ||
| + | :When the procedure that is general for all ratios is repeated on them, a species of the multiple superpartient ratio is revealed, arranged in its kind, according to the arrangement of the given superpartient ratio of its kind, as can be seen here: | ||
| + | |style="text-align:right;"|וכאשר נסובב בהם המעשה אשר הוא כולל לכל היחסים יראה לנו מין מהכפלים המוסיף חלקים מסודרים בסוגו כסדר המוסיף חלקים המונח כסוגו וזה כמו שתראה הנה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color: blue; text-align:center;" | ||
| + | |- | ||
| + | |25||15||9 | ||
|- | |- | ||
| + | |64||24||9 | ||
| + | |} | ||
| | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | |כה||טו||ט | ||
| + | |- | ||
| + | |סד||כד||ט | ||
| + | |} | ||
| + | |- | ||
| + | |It has already become clear that all these ratios that were brought here as an example were produced from the line of the three equal terms, which is the line of the units written in red. | ||
| + | |style="text-align:right;"|הנה כבר התבאר שכל אלו היחסים שהמשלנו אותם בכאן אמנם יתילדו מהטור השוה המדרגות השלשה והוא טור האחדים הנכתבים באודם | ||
| + | |- | ||
| + | |If non-units were placed in this line, as long as they are equal numbers, if we operated on them in the ways we described, then all that we saw of the matters required in the production of these ratios twill necessary follow, except that what will be seen from this is not its cause; rathert its cause is the equality of the units. | ||
| + | |style="text-align:right;"|ואלו הנחנו כמו כן באותו הטור בלתי האחדים רק שיהיו מספרים שוים ועשינו בם מה שעשינו אותו בדרכים אשר תארנו יחוייב שימשך לזה כל מה שנראה לנו מהענינים המתחייבים בהולדת אלו היחסים אלא שאשר נראה מזה אינו עלתו אבל עלתו שווי האחדים | ||
| + | |- | ||
| + | |It can be known that the species of the superpartient ratio produced by this technique are indeed the first of these ratios. | ||
| + | |style="text-align:right;"|וכבר יתכן לנו שנדע כי מיני המוסיף חלקים אשר יתילדו בזאת המלאכה אמנם הם המינים הראשונים מאלו היחסים | ||
| + | |- | ||
| + | |By "the first species" I mean those whose values of their parts exceed the number of their parts by one, as the superbitertian ratio, the supertriquartan ratio, the superquadriquintan ratio, the superquintisextan ratio, and what follows likewise. | ||
| + | |style="text-align:right;"|ורצוני במינים הראשונים מאלו מהם אשר יהיו הגעות חלקיהם יותר מוסיפים ממנין חלקיהם באחד כמו המוסיף שני שלישים ו{{#annot:supertriquartan|128|E9k3}}המוסיף שלשה רביעיות{{#annotend:E9k3}} ו{{#annot:superquadriquintan|128|u8ry}}המוסיף ארבעה חומשים{{#annotend:u8ry}} והמוסיף חמשה שתויות ומה שיעבור זה ממה שידמהו | ||
| + | |- | ||
| + | |The rest of the species of the superpartient ratio, such as the superbiquintan ratio, the supertriquintan ratio, or the supertriseptan ratio, or likewise - the technique for their production is as we stated about in the measure of superpartient ratio. | ||
| + | |style="text-align:right;"|ואמנם שאר מיני המוסיף חלקים כמו המוסיף שני חומשים ו{{#annot:supertriquintan|128|E9gM}}המוסיף שלשה חומשים{{#annotend:E9gM}} או המוסיף שלשה שבעיות או מה שהוא דומה זה הנה המלאכה בהולדתם אמנם היא כמו מה שהגדנו עליה בשעור המוסיף חלקים | ||
| + | |- | ||
| + | |When we want the species of the multiple superpartient ratio in this technique, it is necessary that the given species of the superpartient ratio that will produce it are the first species mentioned, whose value of their parts exceeds the number of their parts by one. | ||
| + | |style="text-align:right;"|וכן גם כן ראוי לנו כשנרצה מיני הכפל המוסיף חלקים בזאת המלאכה שיהיו המינים המונחים מהמוסיף חלקים להוליד זה הם המינים הראשונים אשר זכרנו שהגעת חלקיהם תוסיף על מנין חלקיהם אחד | ||
| + | |- | ||
| + | |This alone is a sufficient discussion of the instruction of whichever species of the multiple superparticular ratio and whichever species of the multiple superpartient ratio that are produced in the mentioned explanation; the rest of the kinds of these ratios are more distant from the way of their kinds, and because of this they are perhaps not visible except by a more complex technique than the one that we have just completed recording. | ||
| + | |style="text-align:right;"|וזה בלבד מאמר מספיק בהוראה על אי זה מיני הכפל המוסיף חלק ואי זה מיני הכפל המוסיף חלקים יתילדו בבאור הנזכר וששאר סוגי אלו היחסים יתרחקו מדרך סוגיהם ולזה אולי לא יראו אלא במלאכה היא יותר רבת ההרכבה מזאת אשר השלמנו לזכרה עתה | ||
| + | |- | ||
| + | |God is the Knower. | ||
| + | |style="text-align:right;"|והאל היודע | ||
| + | |- | ||
| + | |This is for you the conception of the categorization of the relative quantity | ||
| + | |style="text-align:right;"|וזה לך ציור חלוקת הכמה המצטרף | ||
| + | |- | ||
| + | |This, may God lead you straight, is enough for the end of the first section of the book of arithmetic as described by the Pythagorean Nicomachus al-Gehrasīnī. | ||
| + | |style="text-align:right;"|הנה זה יישירך השם מספיק בסוף המאמר הראשון מספר הארתמאיטיקא כפי מה שתיארו ניקמאכוש אלגהרשיני הפתאגורי | ||
| + | |- | ||
| + | |Revised in al-Andalus by Abū Sulaymān Rabīʽ ben Yaḥyā usquf Elvira. | ||
| + | |style="text-align:right;"|ותקנו באנדלס אבו סלימאן רביע בן יחיי אסקף אלבירה | ||
| + | |- | ||
| + | |Find help in its investigation and study | ||
| + | |style="text-align:right;"|והעזר בעיון וההגיה בו | ||
| + | |- | ||
| + | |May God in His mercy lead you straight to understand and find your wishes that are useful for your end, Amen. | ||
| + | |style="text-align:right;"|והאל ברחמיו יישירך להבין ולמצוא חפציך בו המועילים לאחריתך אמן | ||
| + | |- | ||
| + | |The first section of the book of arithmetic is complete. | ||
| + | |style="text-align:right;"|נשלם המאמר הראשון מספר הארתמאטיקא | ||
| + | |- | ||
| + | |Praise be to God. | ||
| + | |style="text-align:right;"|והתהלה לאל | ||
| + | |- | ||
| + | |The second section follows it. | ||
| + | |style="text-align:right;"|יבא אחריו המאמר השני בג"ה | ||
|} | |} | ||
{| | {| | ||
|- | |- | ||
| | | | ||
| + | |||
== Book Two == | == Book Two == | ||
| − | + | |style="text-align:right;"|<big>המאמר השני מספר הארתמאטיקא</big> | |
|- | |- | ||
| | | | ||
| − | === Reducing the Ratios to Equality === | + | === The First Section: Reducing the Ratios to Equality === |
| − | + | |style="text-align:right;"|<big>המאמר הראשון ממנו בהתכת היחסים אל השווי</big> | |
| + | |- | ||
| + | |'''He''' [= Nicomachus] '''said''': the principle and the foundation is the thing from which another thing is composed and into which another thing is decomposed. | ||
| + | |style="text-align:right;"|<big>אמר</big> שהפנה והיסוד הוא הדבר אשר הורכב ממנו דבר אחר ויותך אליו דבר אחר | ||
| + | |- | ||
| + | |For example: | ||
| + | *The alphabet letters, as it is said about them that they are elements of the given writings that are composed of them and decomposed into them. | ||
| + | |style="text-align:right;"|כמו אותיות האלפא ביתא כי יאמר בם שהן פנות לכתבים המונחים המחוברים מהם הנתכים אליהם | ||
| + | |- | ||
| + | | | ||
| + | *The sounds are elements of the melodies, for the melodies are composed from them and decomposed into them. | ||
| + | |style="text-align:right;"|וכן הנעימות הן פנות לכל הלחנים אחר שהיו הלחנים מחוברים מהם נתכים אליהם | ||
| + | |- | ||
| + | | | ||
| + | *The four elements, i.e. the fire, air, water, and earth, are said to be principles and simple elements of for all that is subject to the generation and corruption, for they are composed of them and decomposed into them. | ||
| + | |style="text-align:right;"|וכן גם כן אלו היסודות הארבעה ר"ל האש והאויר והמים והארץ יאמר שהם פנות ופשוטים לכל הנופלים תחת ההויה וההפסד אחר שחבורם מהם והתכתם אליהם | ||
| + | |- | ||
| + | |These propositions were brought forward to demonstrate that equality is a principle of the relative quantity, from which it yields and to which it is reduced. | ||
| + | |style="text-align:right;"|ואמנם הקדמנו אלו ההקדמות להראות שהשווי פנה ל{{#annot:term|107,2579|HKl1}}כמות המצטרף{{#annotend:HKl1}} ושהוא ממנו צמח ואליו יותך | ||
| + | |- | ||
| + | |As for the absolute quantity, its first principle, from which it is produced, and which can be added endlessly, is the one. | ||
| + | |style="text-align:right;"|ואמנם הכמה הנפרד הנה פנתו הראשונה ובו התילד ואיפשר להוסיף בו אל מה שאין תכלית לו הוא אחד | ||
| + | |- | ||
| + | |What is produced from equality, and reduced to it, is common to all the relations. | ||
| + | |style="text-align:right;"|ואמנם מה שיתילד מן השווי ויותך אליו הנה הוא כולל לכל הצרופים | ||
| + | |- | ||
| + | |A proof will be brought, by demonstration and true meaning, that equality is a cause of the relative quantity, from which it is produced, by which it yields when added, and into which it is consumed when subtracted and divided. | ||
| + | |style="text-align:right;"|ונביא ראיה במופת ואמתות שהשווי סבה לכמות המצטרף אשר ממנו היה הולדתו ובו צמח בהתוספו ואליו יכלה כאשר חוסר ונתך | ||
| + | |- | ||
| + | |From the general technique one knows that when setting three numbers, such that the ratio of the first of them to the second is as the ratio of the second to the third [<math>\scriptstyle{\color{blue}{a_1:a_2=a_2:a_3}}</math>], in any species of the five ratios mentioned in the first section, these three given numbers are also such that the ratio of the third to the second is also as the ratio of the second to the first [<math>\scriptstyle{\color{blue}{a_3:a_2=a_2:a_1}}</math>], when the first is the smaller, the second is the mean, and the third is the greater. | ||
| + | |style="text-align:right;"|ומהתחבולה הכוללת לזה הוא שתדע שכאשר תניח שלשה מספרים יחס הראשון מהם אל השני כיחס השני אל השלישי באי זה מהמינים היה מהיחסים החמש אשר זכרנום במאמר הראשון הנה אלו מספרים שלשה אם הונחו כן היה יחס גם כן השלישי אל השני כיחס השני אל הראשון והראשון הוא הקטן והשני הוא האמצעי והשלישי הוא הגדול | ||
| + | |- | ||
| + | | | ||
| + | :If one wishes to restore them to equality: subtract the smaller from the mean, then subtract from the greater the mean and what remained from the mean. [<math>\scriptstyle{\color{blue}{a_3-\left(a_2-a_1\right)-a_2}}</math>] | ||
| + | ::[<math>\scriptstyle{\color{red}{a_1;a_2-a_1;a_3-\left(a_2-a_1\right)-a_2}}</math>] | ||
| + | |style="text-align:right;"|ואם רצה רוצה להשיבם אל השווי הנה ישליך מהאמצעי כמו הקטן עוד ישליך מהגדול כמו מה שישאר מהאמצעי וכמו מהאמצעי | ||
| + | |- | ||
| + | | | ||
| + | :If the three numbers are equalized, it is the required. | ||
| + | |style="text-align:right;"|ואם השתוו המספרים השלשה הנה כבר היה מה שרצינו | ||
| + | |- | ||
| + | | | ||
| + | :If not, they departed from the species of their ratio, and restored to another species anterior by nature to the ratio in which they were first. | ||
| + | |style="text-align:right;"|ואם לא הנה כבר יצאו ממין צרופם ושבו אל מין אחר הוא יותר קודם בטבע ממין היחס אשר היו ממנו ראשונה | ||
| + | |- | ||
| + | | | ||
| + | :Proceed as in the first procedure. | ||
| + | |style="text-align:right;"|עוד תעשה בו כמו המעשה הראשון | ||
| + | |- | ||
| + | | | ||
| + | :If they are equalized, we found what we required. | ||
| + | |style="text-align:right;"|ואם השתוו הנה מצאנו מבוקשנו | ||
| + | |- | ||
| + | | | ||
| + | :If not, they departed from that species also and restored to a species even prior by nature to the first. | ||
| + | |style="text-align:right;"|ואם לא הנה כבר יצאו מאותו המין גם כן אל מין יותר רחוק מן הראשון בטבע | ||
| + | |- | ||
| + | | | ||
| + | :One does not cease to do so, until they are equalized. | ||
| + | |style="text-align:right;"|ולא תסור מעשות כן תמיד עד שישתוו | ||
| + | |- | ||
| + | |Example: | ||
| + | |style="text-align:right;"|ומשל זה | ||
| + | |- | ||
| + | | | ||
| + | *Placing the equality in a line of three ranks. | ||
| + | |style="text-align:right;"|נניח השווי בטור בעל שלשה מדרגות | ||
| + | |- | ||
| + | | | ||
| + | *Arranging a second line of multiple ratio | ||
| + | |style="text-align:right;"|ונסדר אליו טור שני מתיחס ביחס הכפל | ||
| + | |- | ||
| + | | | ||
| + | *Arranging a third line of superparticular ratio | ||
| + | |style="text-align:right;"|עוד נסדר בם טור שלישי על יחס המוסיף חלק | ||
| + | |- | ||
| + | | | ||
| + | *Continuing with a fourth line of superpartient ratio | ||
| + | |style="text-align:right;"|ונמשיך בזה טור רביעי על יחס המוסיף חלקים | ||
| + | |- | ||
| + | | | ||
| + | *Placing in a fifth line numbers that relate in a multiple superparticular ratio | ||
| + | |style="text-align:right;"|עוד נניח בטור החמשי מספרים מתיחסים ביחס הכפל המוסיף חלק | ||
| + | |- | ||
| + | | | ||
| + | *Completing the diagram with a sixth line relating the ranks in a multiple superpartient ratio. | ||
| + | |style="text-align:right;"|ונחתום הצורה בטור ששי מתיחס המדרגות ביחס הכפל המוסיף חלקים | ||
| + | |- | ||
| + | |As in this diagram: | ||
| + | |style="text-align:right;"|כפי מה שבצורה | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color: blue; text-align:center;" | ||
| + | |- | ||
| + | |1||1||1 | ||
| + | |- | ||
| + | |4||2||1 | ||
| + | |- | ||
| + | |9||6||4 | ||
| + | |- | ||
| + | |25||15||9 | ||
| + | |- | ||
| + | |25||10||4 | ||
| + | |- | ||
| + | |64||25||9 | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | |א||א||א | ||
| + | |- | ||
| + | |ד||ב||א | ||
| + | |- | ||
| + | |ט||ו||ד | ||
| + | |- | ||
| + | |כה||טו||ט | ||
| + | |- | ||
| + | |כה||י||ד | ||
| + | |- | ||
| + | |סד||כה||ט | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | :Whichever line that we consider from among these lines, and on which the mentioned reduction operation is done, is restored to the ratio of a line that is closer than it to equality, i.e. closer to the line of the equal terms. | ||
| + | |style="text-align:right;"|הנה זה הטור שנכוין אליו מאלו הטורים ועשינו בו מלאכת ההתכה אשר זכרנוה נשיבהו בזה אל יחס טור הוא יותר קרוב ממנו אל השווי ר"ל שהוא יותר קרוב אל טור הגבולים השוים | ||
| + | |- | ||
| + | | | ||
| + | :One does not cease to operate so, until its ranks are restored to the ratio of the line of equality itself. | ||
| + | |style="text-align:right;"|עוד לא נסור מעשות זה בו עד שישובו מדרגותיו אל יחס טור השווי עצמו | ||
| + | |- | ||
| + | |What was said is enough, as opposed to extending examples and the long repetition that are not adequate for the one who knows that the fulfillment of the arts is not by multitude of examples, but by setting determinations/definitions. | ||
| + | |style="text-align:right;"|ויספיק לך מה שאמרנו מלהרבות ההמשלים וההכפלה הארוכה אשר לא יאותו למי שידע שהשלמת המלאכות לא תהיה ברבוי ההמשלים אבל בתקון הגבולים | ||
| + | |- | ||
| + | |It is now clear that the procedure, which was brought forward in the first section for the production of the ratios, reverses itself when trying to reduce the ratios to equality. | ||
| + | |style="text-align:right;"|וכבר התבאר כי המעשה אשר הקדמנוהו להולדת היחסים במאמר הראשון הוא בעצמו יתהפך הנה כאשר נשתדל להתיך אותם היחסים אל השווי | ||
| + | |- | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | === The Discussion on the Arrangement of the Terms of each Ratio of two Given Numbers from Superparticulars === | ||
| + | |||
| + | |style="text-align:right;"|<big>הדבור בסדור גבולי כל יחס מונח שני המספרים מיחסי המוסיף חלק</big> | ||
| + | |- | ||
| + | |Since we very much need to set successive proportional numbers of the sesquialter ratio, the sesquitertian ratio, the sesquiquartan ratio, or other ratios of the superparticular ratios, we should bring an artful extraction procedure for it and a technique that will verify its assumption, in order that the investigation of what we investigate will be without confusion. | ||
| + | |style="text-align:right;"|וראוי אחר שכבר נצטרך הרבה אל שנניח מספרים נמשכים מתיחסים מהמוסיף חצי או המוסיף שליש או המוסיף רביע או זולת מה מהמוסיף חלק אחר שנביא הוצאה מלאכותית לזה ותחבולה תאמת לנו הסברא בה כדי שתהיה דרישתנו למה שנדרשנו מזה מבלתי בלבול | ||
| + | |- | ||
| + | | | ||
| + | *It is said that the sesquialter ratio is the first of the superparticular ratios, as is visible above. | ||
| + | |style="text-align:right;"|ונאמר שיחס המוסיף חצי הוא ראש {{#annot:term|125,1659|tdFq}}יחסי המוסיף חלק{{#annotend:tdFq}} כמו שנגלה במה שקדם | ||
| + | |- | ||
| + | | | ||
| + | :The first two numbers that produce the sesquialter ratio are two and three. | ||
| + | |style="text-align:right;"|ושתחלת שני מספרים פועלים {{#annot:sesquialter|125|kQ7e}}יחס המוסיף חצי{{#annotend:kQ7e}} הם שנים ושלשה | ||
| + | |- | ||
| + | | | ||
| + | :When wishing to explain how the succeeding terms of this ratio are extracted: | ||
| + | |style="text-align:right;"|וכאשר נרצה לבאר איך נוציא הגבולים הנלוים בזה היחס | ||
| + | |- | ||
| + | | | ||
| + | :*Arranging the ranks of the double ratio, which are the ranks of the even-times-even numbers, in a line | ||
| + | |style="text-align:right;"|נסדר מדרגות הכפל השניי בטור והם מדרגות זוג הזוג | ||
| + | |- | ||
| + | | | ||
| + | ::Leaving out the first rank, since it is one, which has no half. | ||
| + | |style="text-align:right;"|ונשליך המדרגה הראשונה לפי שהיא האחד ואין חצי לו | ||
| + | |- | ||
| + | | | ||
| + | :*Placing beneath each rank the number that produces with it the sesquialter ratio, so a second line is generated that is one rank shorter than the first line, as the corresponding one was removed. | ||
| + | |style="text-align:right;"|עוד נניח תחת כל מדרגה המספר הפועל עמה {{#annot:sesquialter|125|ihTJ}}יחס הדמיון וחצי{{#annotend:ihTJ}} ר"ל יחס המוסיף חצי ויתחדש טור שני חסר מהטור הראשון מדרגה והיא אשר השלכנו בנוכח האחד | ||
| + | |- | ||
| + | | | ||
| + | ::Leaving out again the first rank of this second line, since it is three, which has no half. | ||
| + | |style="text-align:right;"|עוד נשוב ונשליך כמו כן המדרגה הראשונה מזה הטור השני לפי שהוא שלשה ואין חצי לו | ||
| + | |- | ||
| + | | | ||
| + | :*Placing beneath each of its remaining ranks the number that produces with it the sesquialter ratio, so a third line is generated that is one rank shorter than the second line, which is the removed term corresponding to three. | ||
| + | |style="text-align:right;"|ונניח תחת כל אחת ממדרגותיו הנשארות המספר הפועל עמו יחס המוסיף חצי ויתחדש טור שלישי חוסר מהטור השני מדרגה והיא המושלכת נגד השלשה | ||
| + | |- | ||
| + | | | ||
| + | ::Leaving out again the first rank of this third line, since it is nine, which has no half. | ||
| + | |style="text-align:right;"|עוד נשוב גם כן ונשליך המדרגה הראשונה מזה הטור השלישי לפי שהוא תשעה ואין לו חצי | ||
| + | |- | ||
| + | | | ||
| + | :*Placing beneath each of its ranks the number that produces with it the sesquialter ratio, so a fourth line is generated that is one rank shorter than the third line, which is the term removed corresponding the nine. | ||
| + | |style="text-align:right;"|ונניח תחת כל אחת ממדרגותיו המספר הפועל עמו יחס המוסיף חצי הנה יתחדש טור רביעי חוסר מהטור השלישי מדרגה והיא אשר השלכנו כנגד התשעה | ||
| + | |- | ||
| + | | | ||
| + | :*Generating also the fifth and the sixth lines and onwards as is seen in this diagram: | ||
| + | |style="text-align:right;"|וכמו כן נחדש טור חמישי וששי ולמעלה מזה כמו שתראה בזאת הצורה | ||
| + | |- | ||
| + | | | ||
| + | :{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | |32||16||8||4||2||1 | ||
| + | |- | ||
| + | |48||24||12||6||3 | ||
| + | |- | ||
| + | |72||36||18||9 | ||
| + | |- | ||
| + | |108||54||27 | ||
| + | |- | ||
| + | |162||81 | ||
| + | |- | ||
| + | |243 | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | |לב||יו||ח||ד||ב||א | ||
| + | |- | ||
| + | |מח||כד||יב||ו||ג | ||
| + | |- | ||
| + | |עב||לו||יח||ט | ||
| + | |- | ||
| + | |קח||נד||כז | ||
| + | |- | ||
| + | |קסב||פא | ||
| + | |- | ||
| + | |רמג | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | :It is clear that the beginning of the sesquialter ratio is in two terms. | ||
| + | |style="text-align:right;"|וכבר התבאר שתחלת מה שימצא יחס המוסיף חצי בשני גבולים | ||
| + | |- | ||
| + | | | ||
| + | :It is also clear from this diagram how it is found in three, four, five, or six terms. | ||
| + | |style="text-align:right;"|עוד התבאר בזאת הצורה איך נמצאהו בשלשה גבולים עוד בארבעה עוד בחמשה עוד בששה | ||
| + | |- | ||
| + | |Through the technique, by which this diagram is made, terms are added in this ratio endlessly, and no term is missing in any available space in which this technique is not evident. | ||
| + | |style="text-align:right;"|והמלאכה שנעשית בה זאת הצורה כבר יתוספו בה הגבולים בזה היחס אל מה שאין תכלית לו ולא יבצר מהם גבול בשום פנוי שלא תראה בו התחבולה | ||
| + | |- | ||
| + | | | ||
| + | *If we wish to produce numbers according to this example in the sesquitertian ratio: | ||
| + | |style="text-align:right;"|ואם רצינו להוליד מספרים כפי זה המשל ביחס המוסיף חלק השלישי | ||
| + | |- | ||
| + | | | ||
| + | :*Arranging what is in the line of the successive triple ratio: 1; 3; 9; 27; 81; 243. | ||
| + | |style="text-align:right;"|נסדר מה שבטור הכפל השלישי הנמשך על יחס דמיון א' ג' ט' כ"ז פ"א רמ"ג | ||
| + | |- | ||
| + | | | ||
| + | :*Arranging lines beneath this line, placing under each number its likeness plus its third. | ||
| + | |style="text-align:right;"|ונסדר טורים תחת זה הטור נעמיד תחת כל מספר כמהו וכמו שלישיתו מה שאפשר בו זה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*Drawing a diagram, like the first that we drew before, presenting the terms of the sesquitertian ratio without missing any of the terms, as is seen in the following diagram: |
| + | |style="text-align:right;"|ונצייר צורתו כמו מה שציירנו הראשונה אשר לפניה ויראו בה מדרגות הגבולים ב{{#annot:sesquitertian|125|lfra}}יחס המוסיף שליש{{#annotend:lfra}} מבלתי שיחטא בגבול מהם כמו שתראה בזאת הצורה אשר לפניך | ||
|- | |- | ||
| | | | ||
| − | == | + | :{|class="wikitable" style="color:blue; text-align:center;" |
| + | |- | ||
| + | |243||81||27||9||3||1 | ||
| + | |- | ||
| + | |324||108||36||12||4 | ||
| + | |- | ||
| + | |432||144||48||16 | ||
| + | |- | ||
| + | |576||192||64 | ||
| + | |- | ||
| + | |768||256 | ||
| + | |- | ||
| + | |1024 | ||
| + | |} | ||
| − | + | | | |
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | |רמג||פא||כז||ט||ג||א | ||
| + | |- | ||
| + | |שכד||קח||לו||יב||ד | ||
| + | |- | ||
| + | |תלב||קמד||מח||יו | ||
| + | |- | ||
| + | |תקעו||קצב||סד | ||
| + | |- | ||
| + | |תשסח||רנו | ||
| + | |- | ||
| + | |אלף כד | ||
| + | |} | ||
| + | |||
| + | |- | ||
| + | | | ||
| + | *Likewise when we want the sesquiquartan ratio: 1; 4; 16; 64 | ||
| + | |style="text-align:right;"|וכמו כן כאשר נרצה ב{{#annot:sesquiquartan|125|Yooq}}יחס המוסיף חלק הרביעיי{{#annotend:Yooq}} הנמשך על יחס דמיון א' ד' י"ו ס"ד | ||
| + | |- | ||
| + | | | ||
| + | *Also for any species of the soecies of the superparticular ratios that follow. | ||
| + | |style="text-align:right;"|וכמו כן מה שילוה לזה מין ממיני המוסיף חלק | ||
| + | |- | ||
| + | |The basis of the technique of this diagram is to place the successive terms of the first line in the multiple ratio, after which the superparticular ratio of the required terms is nameed, i.e. the multiple that yields the denominator of the part. | ||
| + | |style="text-align:right;"|ואמנם היסוד במלאכת זאת הצורה שנשים מדרגות הטור הראשון נמשכים על יחס הכפל אשר הוא נקרא ליחס הדרוש הגבולים מיחסי המוסיף חלק ר"ל הכפל אשר ממנו מעמד אותו החלק | ||
| + | |- | ||
| + | |When it is produced in this diagram by the mentioned technique, it is evident and clear that the numbers that are placed in the angles [the main diagonal] of each diagram are the numbers that are in the first given line of the following diagram. | ||
| + | |style="text-align:right;"|וכאשר עשית אותו מזאת הצורה במלאכת הנזכרת הוא גלוי מבואר שהמספרים אשר יפלו בזויות מכל צורה הם המספרים אשר בטור הראשון המונח בצורה אשר תמשך לה | ||
| + | |- | ||
| + | |Here are other notable and wonderful properties that you will understand through your good examination by the will of God. | ||
| + | |style="text-align:right;"|ובכאן עניינים אחרים נכבדים ונפלאים תעמוד עליהם עם טוב בחינתך בהם ברצון השם | ||
| + | |- | ||
| + | |Revealing which ratio of the superparticular ratios is found in a composition, i.e. from the composition of which of the ratios follows whichever multiple ratio required. | ||
| + | |style="text-align:right;"|על גלוי אי זה יחס מיחסי המוסיף חלק הנמצא בחבור ר"ל נמצא בחבוריהם יחס מונח ופי' איזה יחסים הם אשר יתחייב מחבורם יחס איזה כפל שתדרוש בחבור יחס מונח מיחסי בעל הכפלים | ||
| + | |- | ||
| + | | | ||
| + | *The first species of the multiple ratios, which is the double ratio, is composed and produced through the first two types of the superparticular ratios, which are the sesquialter ratio and the sesquitertian ratio. | ||
| + | |style="text-align:right;"|אמנם המין הראשון מבעל הכפלים והוא השניי הנה הוא יתחבר ויתילד באמצעות שני המינים הראשונים ממיני המוסיף חלק והם המוסיף חצי והמוסיף שליש | ||
| + | |- | ||
| + | | | ||
| + | ::For example: | ||
| + | ::*<math>\scriptstyle{\color{blue}{4=3+\left(\frac{1}{3}\sdot3\right)}}</math> | ||
| + | |style="text-align:right;"|משל זה שהארבעה כמו השלשה וכמו שלישיתם | ||
| + | |- | ||
| + | | | ||
| + | ::*<math>\scriptstyle{\color{blue}{3=2+\left(\frac{1}{2}\sdot2\right)}}</math> | ||
| + | |style="text-align:right;"|והשלשה כמו השנים וכמו חציו | ||
| + | |- | ||
| + | | | ||
| + | :*<math>\scriptstyle{\color{blue}{4:2}}</math> in the double ratio, as one sees in this diagram. | ||
| + | |style="text-align:right;"|והנה הארבעה אצל השנים ביחס הכפל השניי כמו שתראה בזאת הצורה | ||
| + | |- | ||
| + | | | ||
| + | :By the converse explanation it is clear that the double ratio is dissolved to the sesquialter ratio and the sesquitertian ratio. | ||
| + | |style="text-align:right;"|ובהפך זה המאמר יתבאר שהכפל השניי יותך אל המוסיף חצי והמוסיף שליש | ||
| + | |- | ||
| + | | | ||
| + | ::For example: if we take two numbers in the double ratio, such as 6 and 3, we will find a mean number between them, by which the double ratio of the two given extremes is dissolved to the two mentioned ratios of the superparticular ratios. | ||
| + | |style="text-align:right;"|משל זה שאם לקחנו שני מספרים ביחס הכפל השניי כמו הששה והשלשה שאנו נמצא ביניהם אמצעי מן המספר יותך אצלו יחס שני הקצוות המונחים מהכפל השניי על שני היחסים הנזכרים מיחסי המוסיף ואם כן יותך אליהם חלק | ||
| + | |- | ||
| + | | | ||
| + | ::The mean between 6 and 3 is 4: | ||
| + | |style="text-align:right;"|והאמצעי בין הששה והשלשה הם ארבעה | ||
| + | |- | ||
| + | | | ||
| + | ::*<math>\scriptstyle{\color{blue}{6:4}}</math> sesquialter ratio | ||
| + | |style="text-align:right;"|הנה הששה כאשר הוקשו אל הארבעה היה המוסיף חצי | ||
| + | |- | ||
| + | | | ||
| + | ::*<math>\scriptstyle{\color{blue}{4:3}}</math> sesquitertian ratio | ||
| + | |style="text-align:right;"|והארבעה אצל השלשה תוסיף שליש | ||
| + | |- | ||
| + | | | ||
| + | :And when it is dissolved, it is dissolved to them. | ||
| + | |style="text-align:right;"|וכאשר יותך אמנם יותך אליהם | ||
| + | |- | ||
| + | | | ||
| + | *The second type of the multiple ratios, i.e. the triple ratio, is produced from the first type of the superparticular ratios, i.e. the sesquialter ratio, and the first type of the multiple ratios, i.e. the double ratio. | ||
| + | |style="text-align:right;"|ואמנם המין השני מבעל הכפלים ר"ל הכפל השלישיי אמנם יולד מהמין הראשון המוסיף חלק ר"ל החצי והמין הראשון מבעל הכפלים ר"ל השניי | ||
| + | |- | ||
| + | | | ||
| + | ::For example: | ||
| + | ::*<math>\scriptstyle{\color{blue}{18=12+\left(\frac{1}{2}\sdot12\right)}}</math> | ||
| + | |style="text-align:right;"|משל זה שי"ח כמו י"ב וכמו חציו | ||
| + | |- | ||
| + | | | ||
| + | ::*<math>\scriptstyle{\color{blue}{12=2\sdot6}}</math> | ||
| + | |style="text-align:right;"|וי"ב כפל ו‫' | ||
| + | |- | ||
| + | | | ||
| + | ::*<math>\scriptstyle{\color{blue}{18:6=3}}</math> triple ratio | ||
| + | |style="text-align:right;"|אבל י"ח אצל ו' כיחס הכפל השלישיי | ||
| + | |- | ||
| + | | | ||
| + | ::You will find the same again, except that it is not necessary to set 12, which is double 6, as the mean, but we may set 9 instead, which is like 6 plus its half. | ||
| + | ::*<math>\scriptstyle{\color{blue}{9=6+\left(\frac{1}{2}\sdot6\right)}}</math> | ||
| + | |style="text-align:right;"|וכמו כן גם כן תמצאהו אלא שלא יחוייב שנשים האמצעי י"ב אשר הוא כפל ו' אבל נשים במקומו ט' אשר הוא כמו ו' וכמו חציו | ||
| + | |- | ||
| + | | | ||
| + | ::Both forms are already demonstrated. | ||
| + | |style="text-align:right;"|וכבר המשלתי לך שתי הצורות | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :By the converse explanation it is clear that the triple ratio, when it is dissolved, dissolves into the double ratio and the sesquialter ratio. |
| + | |style="text-align:right;"|ובהפך זה המאמר יתבאר שהכפל השלישי כאשר יותך אמנם יותך אל הכפל השניי והמוסיף חצי | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|וכלל המאמר שכל מין ממיני הכפל כאשר הורכב עם דומה לו במדרגה ממיני המוסיף חלק הנה יתחבר מהם המין אשר ילוה אליו בקרוב שבמדרגות אליהם | + | ::Examine it with whichever of the two numbers you wish by setting them in the triple ratio, and you will find it so. |
| + | |style="text-align:right;"|ובחן זה באי זה משני מספרים תרצה אם תניחם ביחס הכפל השלישיי ותמצאהו כן | ||
| + | |- | ||
| + | | | ||
| + | *If the triple ratio and the second type of the superparticular ratio are composed, the quadruple ratio is produced. | ||
| + | |style="text-align:right;"|ואמנם אם יחובר הכפל השלישיי והמין השני מהמוסיף חלק יתילד מזה הכפל הרביעיי | ||
| + | |- | ||
| + | | | ||
| + | :And if the quadruple ratio is dissolved, it dissolves into the triple ratio and to the second type of the superparticular ratio. | ||
| + | |style="text-align:right;"|וכאשר יותך הכפל הרביעיי הנה הוא יותך אל הכפל השלישיי ואל {{#annot:sesquialter|125|izhT}}המוסיף חלק השניי{{#annotend:izhT}} | ||
| + | |- | ||
| + | |The rule of the discussion is that every species of the multiple ratios, when it is composed with its corresponding species of the superparticular ratios, the closest type that follows it among the multiple ratios is composed from them. | ||
| + | |style="text-align:right;"|וכלל המאמר שכל מין ממיני הכפל כאשר הורכב עם דומה לו במדרגה ממיני המוסיף חלק הנה יתחבר מהם המין אשר ילוה אליו בקרוב שבמדרגות אליהם ממיני הכפל | ||
| + | |- | ||
| + | |This is the full discussion on the relative quantity with all the aspects of its relations, while keeping as much as possible the completion of the properties by a brief discussion about that which the scholars cannot do without. | ||
| + | |style="text-align:right;"|זהו כלל המאמר בכמה המצטרף על כל צדי צירופיו עם תכלית מה שאיפשר מהשמירה בהשלמת הענינים עם קצור המאמר במה שאי אפשר למתלמדים בלעדיו | ||
| + | |} | ||
| + | {| | ||
|- | |- | ||
| | | | ||
| − | === Absolute Quantity === | + | |
| + | === [Absolute Quantity] === | ||
| | | | ||
|- | |- | ||
| | | | ||
| − | ==== Numbers | + | ==== The Discussion of the Quality of the Absolute Numbers that are Potentially Commensurable with the Geometric Shapes ==== |
| − | + | |style="text-align:right;"|<big>הדבור בתואר המספרים הנפרדים המשותפים בכחם לתמונות המידותיות</big> | |
| + | |- | ||
| + | |The beginning of this discussion on the geometrical shapes: | ||
| + | |style="text-align:right;"|ותחלת זה הדבור בתמוניות | ||
| + | |- | ||
| + | |Now we note what remains of the things that belong to the absolute quantity, which requires a more intensive investigation than all that we discussed in the first section. | ||
| + | |style="text-align:right;"|אמנם עתה הנה אנחנו זוכרים מה שנשאר מהדברים המתחייבים לכמה הנפרד ממה שיצטרך לחקירה יותר חזקה מכל מה שדברנו עליו במאמר הראשון | ||
| + | |- | ||
| + | |Indeed, when we wish to note it, there is something that we need in order to derive evidence concerning another. | ||
| + | |style="text-align:right;"|כי כאשר אנחנו רוצים לזכרו הנה הוא דבר נצטרך אליו לקחת ממנו ראיה על זולתו | ||
| + | |- | ||
| + | |The wise men used to praise the explanation of some sciences through others. | ||
| + | |style="text-align:right;"|והחכמים היו משבחים מאד לפרש קצת החכמות בקצת | ||
| + | |- | ||
| + | |Of the things whose explanation should be prefaced, it should be said that: | ||
| + | |style="text-align:right;"|ומהדברים אשר ראוי להקדים בבאורם שנאמר כי | ||
| + | |- | ||
| + | | | ||
| + | *Some of the numbers are similar to lines in their arrangement. | ||
| + | |style="text-align:right;"|מהמספרים מה שהוא דומה לקוים בסדרם | ||
| + | |- | ||
| + | | | ||
| + | *Some of them are similar to a surface. | ||
| + | |style="text-align:right;"|ומהם מה שהוא דומה לשטח | ||
| + | |- | ||
| + | | | ||
| + | *Some of them are similar to a cube. | ||
| + | |style="text-align:right;"|ומהם מה שהוא דומה למעוקב | ||
| + | |- | ||
| + | | | ||
| + | *Some of them are similar to a sphere. | ||
| + | |style="text-align:right;"|ומהם מה שהוא דומה לכדור | ||
| + | |- | ||
| + | | | ||
| + | *Some of them are similar to a brick in the deficiency of the height with respect to the length and width. | ||
| + | |style="text-align:right;"|ומהם מה שהוא דומה ללבנה בחסרון הגובה מהאורך והרחב | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *Some of them are similar to a pillar in the deficiency of the length and width with respect to the height. |
| + | |style="text-align:right;"|ומהם מה שהוא דומה לעמוד בחסרון האורך והרחב מהגובה | ||
|- | |- | ||
| | | | ||
| − | ===== | + | *Some of them are similar to an arc. |
| + | |style="text-align:right;"|ומהם מה שהוא דומה לקשת | ||
| + | |- | ||
| + | |Likewise for each of the things of geometry, i.e. of measure - these shapes have a likeness in numbers. | ||
| + | |style="text-align:right;"|וכמו כן לכל אחד מהדברים אשר להנדסה ר"ל המדות יש דמיון לאותם התמונות במספרים | ||
| + | |- | ||
| + | |For geometry is based on quality more than on quantity. | ||
| + | |style="text-align:right;"|כי ההנדסה לאיכות יותר דבקה מאשר לכמות | ||
| + | |- | ||
| + | |Look carefully on what was mentioned about the precedence of the number over the measures in the introduction to the number, because it was noticed and discussed there. | ||
| + | |style="text-align:right;"|ותעיין מה שזכרנו מקדימת המספר למדות בפתיחת המספר כי שם שמנו אליו לב ודברנו בו | ||
| + | |- | ||
| + | |Before discussing the similarity of these mentioned determinations to the numbers, we say that the meaning of the alphabet letters as designating the numbers that are marked by them, which are the shapes that are seen, which the people call decimal calculation, is not in their nature, nor in their law, but they designate them only by convention and positing. | ||
| + | |style="text-align:right;"|וקודם שנדבר על דמיון אלו הגבולים אשר זכרנום מהמספרים נאמר שהוראת אותיות האלפא ביתא על מה שיורו עליו מן המספרים הנרשמים בם והם הרשמים אשר אנו רואים שיקראום האנשים חשבון הכללים אינו בטבע להם ולא בדין להם כך אבל הם מורים בהסכמה ובהנחה ממנו לא זולתו | ||
| + | |- | ||
| + | |Those [sign] that indicate [numbers] by nature are like those [objects] visible by the sense. | ||
| + | |style="text-align:right;"|ואמנם המורים בטבעם הנה הם כמו הנראים לחוש | ||
| + | |- | ||
| + | | | ||
| + | *If we wish to write one - we set one sign. | ||
| + | |style="text-align:right;"|והוא שאנו כאשר נרצה לרשום אחד נניח סימן אחד | ||
| + | |- | ||
| + | | | ||
| + | *If we wish to write two - we set two signs. | ||
| + | |style="text-align:right;"|וכשנרצה לרשום שנים נניח שני סמנים | ||
| + | |- | ||
| + | | | ||
| + | *If we wish [to write] three - we set three signs. | ||
| + | |style="text-align:right;"|וכן אם נרצה שלשה נניח שלשה סמנים | ||
| + | |- | ||
| + | |These will be the form of the one, the form of the two, the form of the three, and so on for what follows. | ||
| + | |style="text-align:right;"|ותהיה צורת האחד וצורת השנים וצורת השלשה וכן מה שאחר זה | ||
| + | |- | ||
| + | |When we bring this condition, we say that the one is assumed in this craft as the beginning of number, like the rank of the point in the science of geometry, which is the beginning of the line, while the point or the one are neither a line nor a number. | ||
| + | |style="text-align:right;"|וכאשר הבאנו זה התנאי נאמר שאנו נניח האחד בזאת המלאכה התחלה למספר כמדרגת הנקודה במלאכת המדות אשר היא התחלת הקו מבלתי שתהיה הנקודה או האחד קו או מספר | ||
| + | |- | ||
| + | |As when the point is multiplied by itself, no other quantity is generated. | ||
| + | |style="text-align:right;"|הנה כמו שהנקדה כאשר נכפלה בעצמה לא יתחדש גודל אחר | ||
| + | |- | ||
| + | |Because, when what has no magnitude is multiplied by what has no magnitude, no magnitude is generated | ||
| + | |style="text-align:right;"|שמה שאין גודל לו כאשר נכפל במה שאין גודל לו לא יתחדש גודל | ||
| + | |- | ||
| + | |Since no being emerges from non-being. | ||
| + | |style="text-align:right;"|לפי שלא יהיה יש מלא יש | ||
| + | |- | ||
| + | |Likewise the one, which is not a number, when it is multiplied by itself, no number is generated, as no being is generated from the multiplication of nothing. | ||
| + | |style="text-align:right;"|כמו כן האחד אשר אינו מספר כאשר נכפל בעצמו לא יתחדש מספר לפי שלא יתחדש יש מכפל אין | ||
| + | |- | ||
| + | |Hence, the essence of the one, as it is said, is that it is the beginning of the number, but it is not a number. | ||
| + | |style="text-align:right;"|הנה אם כן אמתת האחד כמו שאמרנו שהוא תחלת המספר ואינו מספר | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | ===== Plane Numbers ===== | ||
| + | |||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | ====== The Discussion of the Aspect of Similarity between the Number to the Line ====== | ||
| − | + | |style="text-align:right;"|<big>הדבור בצד התדמות המספר לקו</big> | |
| + | |- | ||
| + | |The beginning of its continuity is found in duplicity. | ||
| + | |style="text-align:right;"|ואמנם תחלת {{#annot:term|2601,2574|I0sK}}המשכותו{{#annotend:I0sK}} אמנם ימצא בשניות | ||
| + | |- | ||
| + | |Furthermore, it increases continually at the rate of the addition of the natural numbers and their production. | ||
| + | |style="text-align:right;"|עוד יתרבה בהמשכות כפי שעור הוספת המספרים הטבעיים וצמיחתם | ||
| + | |- | ||
| + | |As the number is generated by assuming two different units such that the number arises from their difference, so the line is generated by assuming two different point, such that the line arises is from their difference. | ||
| + | |style="text-align:right;"|הנה כמו שהמספר יתחדש בהנחת שני אחדים נבדלים ויהיה מהבדלם המספר כן הקו אמנם יתחדש בהנחת שתי {{#annot:term|833,1606|u4ba}}נקודות{{#annotend:u4ba}} נבדלות הנה יהיה מהבדלם הקו | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:definition|592,1450|mITP}}The line has one dimension. | ||
| + | |style="text-align:right;"|והקו בעל משך אחד{{#annotend:mITP}} | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:definition|814,1310|B67O}}The two-dimensional is the surface. | ||
| + | |style="text-align:right;"|ומה שהיה בעל שני המשכים הוא שטח{{#annotend:B67O}} | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:definition|587,1850|Sy4l}}The three-dimensional is the solid. | ||
| + | |style="text-align:right;"|ומה שהיה בעל שלשה המשכים הוא גשם{{#annotend:Sy4l}} | ||
| + | |- | ||
| + | |The three-dimensional has six directions, which are said to be necessary in every solid, i.e. up, down, right, left, forward, backward. | ||
| + | |style="text-align:right;"|ולבעל השלשה המשכים מה שיתחדש השש פאות אשר יאמר שהן מתחייבות לכל גשם ר"ל מעלה ומטה וימין ושמאל ופנים ואחור | ||
| + | |- | ||
| + | |By these six directions the motion divides the places. | ||
| + | |style="text-align:right;"|ובאלו השש פאות תחלק התנועה המקומות | ||
| + | |- | ||
| + | |For it holds necessarily that each dimension has two opposite directions, whether one has the two directions up and down, or another forward and backward, or another right and left. | ||
| + | |style="text-align:right;"|כי בהכרח שיתחייב לכל משך שתי תכליות מקבילים אם לאחד משתי התכליות מעלה ומטה ואם לאחר פנים ואחור ואם לאחר ימין ושמאל | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:definition|587,1850|nUtW}}One may argue by saying in a general statement that any thing that has length, breadth, and depth is a solid, and every solid has length, breadth, and depth. | ||
| + | |style="text-align:right;"|ואולי יהיה לטוען שיאמר הנה במאמר כולל שכל בעל אורך ורוחב ועומק הוא גשם וכל גשם בעל אורך ורחב ועומק{{#annotend:nUtW}} | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:definition|814,1310|QKfY}}Likewise, one may say that what has only length and breadth is a surface, and the surface is that which has only length and breadth. | ||
| + | |style="text-align:right;"|וכמו כן יאמר מה שהיה לו אורך ורחב לבד הוא שטח והשטח הוא מה שהיה לו אורך ורחב לבד{{#annotend:QKfY}} | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:definition|592,1450|3xJt}}The line is that which has only length, and that which has only length is a line. | ||
| + | |style="text-align:right;"|והקו מה שהיה לו אורך לבד ומה שהיה לו אורך לבד הוא קו{{#annotend:3xJt}} | ||
| + | |- | ||
| + | |Hence: | ||
| + | *The surface is one dimension less than the solid. | ||
| + | |style="text-align:right;"|הנה השטח חסר מהגשם במשך אחד | ||
| + | |- | ||
| + | | | ||
| + | *The line is one dimension less than the surface. | ||
| + | |style="text-align:right;"|והקו מהשטח במשך אחד | ||
| + | |- | ||
| + | | | ||
| + | *The point is one dimension less than the line. | ||
| + | |style="text-align:right;"|והנקודה חסרה מהקו במשך אחד | ||
| + | |- | ||
| + | |Hence, the point has no dimension at all. | ||
| + | |style="text-align:right;"|הנה אם כן ה{{#annot:term|833,1606|Qk2e}}נקודה{{#annotend:Qk2e}} אין מרחק לה כלל | ||
| + | |- | ||
| + | | | ||
| + | *It is the beginning of the line, but it is not a line. | ||
| + | |style="text-align:right;"|ואמנם היא התחלת הקו ואיננה קו | ||
| + | |- | ||
| + | | | ||
| + | *The line is the beginning of the surface, but it is not a surface. | ||
| + | |style="text-align:right;"|וכמו כן ה{{#annot:term|592,1450|RC38}}קו{{#annotend:RC38}} התחלת השטח ואיננו שטח | ||
| + | |- | ||
| + | | | ||
| + | :It is the beginning of the two dimensions, but it is not two-dimensional. | ||
| + | |style="text-align:right;"|והתחלת שני המשכים ואיננו שני המשכים | ||
| + | |- | ||
| + | | | ||
| + | *The surface is the beginning of the solid, but it is not a solid. | ||
| + | |style="text-align:right;"|וכמו כן ה{{#annot:term|814,1310|Lfgr}}שטח{{#annotend:Lfgr}} התחלת ה{{#annot:term|587,1850|Q8TU}}גשם{{#annotend:Q8TU}} ואיננו גשם | ||
| + | |- | ||
| + | | | ||
| + | :It is the beginning of the three dimensions. | ||
| + | |style="text-align:right;"|והתחלת שלשה המשכים | ||
| + | |- | ||
| + | |Thus, the numbers are also similar to these determinations, as will be described. | ||
| + | |style="text-align:right;"|הנה אכן גם כן המספרים הם דומים לאלו הגבולים כפי מה שאתאר | ||
| + | |- | ||
| + | |{{#annot:definition|2462,2101|OyFF}}Indeed, the linear numbers are all those that are set to begin with two and increase by one according to the order of the natural succession. | ||
| + | |style="text-align:right;"|וזה שהמספרים הקויים הם כל מה שתניח התחלתו מהשנים עוד יתרבו באחד על סדר משך הטבע{{#annotend:OyFF}} | ||
| + | |} | ||
| + | {| | ||
|- | |- | ||
| | | | ||
| − | |||
| + | ====== The Discussion of the Triangle as the Foundation of all Rectilinear Surfaces ====== | ||
| + | |style="text-align:right;"|<big>הדבור באשר המשולש יסוד לכל השטחים ישרי הקוים</big> | ||
| + | |- | ||
| + | |The first of the rectilinear surfaces is the triangle. | ||
| + | |style="text-align:right;"|ואמנם {{#annot:term|1141,2568|SU8c}}השטחים ישרי הקוים{{#annotend:SU8c}} הראשון מהם המשולש | ||
| + | |- | ||
| + | |It is the one that has three angles. | ||
| + | |style="text-align:right;"|והוא בעל השלש זויות | ||
| + | |- | ||
| + | |It is as a root and a beginning of all flat surfaces, such as the square, the pentagon, the hexagon, and their similar. | ||
| + | |style="text-align:right;"|והוא כשרש וכהתחלה לכל {{#annot:term|1141,1847|9SO5}}השטחים הישרים{{#annotend:9SO5}} כמו ה{{#annot:term|305,1263|d8ge}}מרובע{{#annotend:d8ge}} וה{{#annot:term|2170,1672|m2Dh}}מחומש{{#annotend:m2Dh}} והמשושה והדומה להם | ||
| + | |- | ||
| + | |For all of them are decomposeable into the triangle. | ||
| + | |style="text-align:right;"|כי כלם יותכו אל המשולש | ||
| + | |- | ||
| + | |But the triangle does not decompose into anything but its likeness. | ||
| + | |style="text-align:right;"|וזה המשולש לא יותך אל בלתי תמונתו | ||
| + | |- | ||
| + | |This is clear, because when you draw lines from the angles of whichever regular shape you wish to its center, the shape, whichever it may be, will be divided into triangles. | ||
| + | |style="text-align:right;"|וזה מבואר מאשר כשתוציא מאיזה מהתמונות הישרות הקוים שתרצה קוים ישרים מזויותיהם אל מרכזה תחלק איזו תמונה שתהיה אל המשולשים | ||
| + | |- | ||
| + | |you find that the triangle also divides itslef into itself, unchanged by its nature and not deviating from its genus. | ||
| + | |style="text-align:right;"|ותמצא המשולש יחלק גם כן עצמו אל עצמו בלתי נבדל מטבעו ולא יצא מסוגו | ||
| + | |- | ||
| + | |It is already visible to the sense from this figure that the triangle, the square, the pentagon, and the rectilinear shapes that follow them, when we draw in them the described lines from their centers to their angles, all of them decompose into triangles. | ||
| + | |style="text-align:right;"|וכבר תראה זה בזאת הצורה לחוש שהמשולש והמרובע והמחומש ומה שאחריהם מהתמונות הישרות הקוים כאשר הוצאנו בם ממרכזיהם אל זויותיהם הקוים אשר תארנו יותכו כלם אל משולשים | ||
| + | |- | ||
| + | |It has now been clarified that the triangle is a root and a beginning of all the shapes. | ||
| + | |style="text-align:right;"|הנה כבר התבאר שהמשולש שרש והתחלה לכל התמונות כלן | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ====== The Discussion of the Triangular Numbers, their Production and their Sides ====== | ||
| + | |||
| + | |style="text-align:right;"|<big>הדבור במשולשים המספריים וצמיחתם וצלעותיהם</big> | ||
| + | |- | ||
| + | |The triangular numbers are sums of the natural numbers beginning from the one. | ||
| + | |style="text-align:right;"|ואמנם המשולשים הם {{#annot:term|388,2511|0Mtq}}הוספות{{#annotend:0Mtq}} המספרים הטבעיים אשר התחלתם האחד קצתם על קצת | ||
| + | |- | ||
| + | | | ||
| + | *The one, when it is written, is a triangle in potentia. | ||
| + | |style="text-align:right;"|הנה האחד כאשר נרשם הנה הוא משולש בכח | ||
| + | |- | ||
| + | | | ||
| + | *When two is added to the one, the three are the beginning of the triangles in actu. <math>\scriptstyle{\color{blue}{1+2=3}}</math> | ||
| + | |style="text-align:right;"|וכאשר נוסיף על האחד השנים היו השלשה ראשית המשולשים בפעל | ||
|- | |- | ||
| | | | ||
| + | *When adding three, the successor of two, to the first actual triangle the result is the second actual triangle, which is 6. <math>\scriptstyle{\color{blue}{1+2+3=3+3=6}}</math> | ||
| + | |style="text-align:right;"|עוד תוסיף שלשה אשר ימשכו לשנים על המשולש הראשון בפעל ויהיה המשולש השני בפעל והוא ששה | ||
| + | |- | ||
| + | | | ||
| + | *When adding four the result is the third actual triangle, which is 10. <math>\scriptstyle{\color{blue}{1+2+3+4=6+4=10}}</math> | ||
| + | |style="text-align:right;"|עוד תוסיף ארבעה ויהיה המשולש השלישי בפעל והוא עשרה | ||
| + | |- | ||
| + | |So on, the production of the triangles is generated from the addition of the natural numbers to each other. | ||
| + | |style="text-align:right;"|עוד כן תתחדש תולדת המשלשים בתוספת המספרים הטבעיים קצתם אל קצתם | ||
| + | |- | ||
| + | |From what you observe in this diagram, the triangular numbers are produced from the addition of the natural numbers to each other. | ||
| + | |style="text-align:right;"|וממה שתבחנהו בזאת הצורה שהמספרים המשולשים יתילדו מחבור המספרים הטבעיים קצתם על קצת | ||
| + | |- | ||
| + | | | ||
| + | ::Such as 3 that bears the composition of 2 and 1 <math>\scriptstyle{\color{blue}{3=2+1}}</math> | ||
| + | |style="text-align:right;"|כמו ג' שתשא חבור ב' וא‫' | ||
| + | |- | ||
| + | | | ||
| + | ::1, 3, 6, 10, 15, 21 | ||
| + | |style="text-align:right;"|א' ג' ו' י' ט"ו כ"א | ||
| + | |- | ||
| + | |The production of the sides of these triangles is as the production of the natural numbers according to their succession: | ||
| + | |style="text-align:right;"|ואמנם התילד צלעות אלו המשולשים הוא כפי התילד המספרים הטבעיים על המשכם | ||
| + | |- | ||
| + | | | ||
| + | *The side of the first in potentia is 1. | ||
| + | |style="text-align:right;"|הנה יהיה צלע הראשון בכח האחד | ||
| + | |- | ||
| + | | | ||
| + | *The side of the first in actu is 2. | ||
| + | |style="text-align:right;"|וצלע הראשון בפעל ב‫' | ||
| + | |- | ||
| + | | | ||
| + | *The side of the second in actu is 3. | ||
| + | |style="text-align:right;"|וצלע השני בפעל ג‫' | ||
| + | |- | ||
| + | | | ||
| + | *The side of the third in actu is 4. | ||
| + | |style="text-align:right;"|וצלע השלישי בפעל ד‫' | ||
| + | |- | ||
| + | |In this way, each side of the triangles is produced from the production of the natural numbers. | ||
| + | |style="text-align:right;"|ובזה הצד מהתילד המספרים הטבעיים יתילד כל צלע מצלעות המשולשים | ||
| + | |- | ||
| + | | | ||
| + | *Beginning from placing the one first. | ||
| + | |style="text-align:right;"|וזה כאשר נתחיל להניח האחד תחלה | ||
| + | |- | ||
| + | | | ||
| + | *Then, each number of the natural numbers is produced by itself in the successive lines beneath the one, as seen illustrated in this diagram: | ||
| + | |style="text-align:right;"|עוד יתילד כל מספר מהמספרים הטבעיים בפני עצמו בטורים ימשכו קצתם לקצת תחת האחד כפי מה שתראה מצוייר בזאת הצורה | ||
| + | |- | ||
| + | | | ||
| + | |[[File:Triangular Numbers.png|thumb|300px|right]] | ||
| + | |- | ||
| + | | | ||
| + | *The first potential triangle is 1, and its side is 1. | ||
| + | |style="text-align:right;"|המשולש הראשון בכח אחד וצלעו אחד | ||
| + | |- | ||
| + | | | ||
| + | *The first actual triangle is 3, and its side is 2. | ||
| + | |style="text-align:right;"|והמשולש הראשון בפעל שלשה וצלעו השני‫' | ||
| + | |- | ||
| + | | | ||
| + | *The second actual triangle is 6, and its side is 3. | ||
| + | |style="text-align:right;"|והמשלש השני בפועל ששה וצלעו שלשה | ||
| + | |- | ||
| + | | | ||
| + | *The third actual triangle is 10, and its side is 4. | ||
| + | |style="text-align:right;"|והמשלש השלישי בפעל עשרה וצלעו ארבעה | ||
| + | |- | ||
| + | | | ||
| + | *The fourth actual triangle is 15, and its side is 5. | ||
| + | |style="text-align:right;"|והמשלש הרביעי בפעל ט"ו וצלעו חמשה | ||
| + | |- | ||
| + | |What should be examined concerning these triangular numbers is that each triangle among them encompasses the geometrical triangles by a number that is equal to the number of the preceding square, i.e. preceding by one rank, and so on always. | ||
| + | |style="text-align:right;"|ומה שראוי שתבחנהו באלו המספרים המשולשים שכל משולש מהם יקיף מהמשלשים המדותיים במניין שוה למספר המרובע הקודם ר"ל במדרגה אחת וכן תמיד | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ====== The Discussion of the Square Numbers, their Sides and their Production ====== | ||
| + | |||
| + | |style="text-align:right;"|<big>הדבור במרובעים המספריים וצלעותיהם והתילדם</big> | ||
| + | |- | ||
| + | |The regular square shapes are not produced as the triangles are produced from the three. | ||
| + | |style="text-align:right;"|ואמנם התמונות המרובעות הישרות לא יתילדו כמו שיתילדו המשולשות מן השלשה | ||
| + | |- | ||
| + | |This is because they have four sides and four angles. | ||
| + | |style="text-align:right;"|וזה שהן בעלות ארבע צלעות וארבע זויות | ||
| + | |- | ||
| + | |Such as: 4; 9; 16; 25; 36 | ||
| + | |style="text-align:right;"|כמו ד' ט' י"ו כ"ה ל"ו | ||
| + | |- | ||
| + | |The shapes of these numbers are quadruple and equilateral, according to this example, i.e. the one illustrated at the end of the chapter, which is the diagram of all regular squares. | ||
| + | |style="text-align:right;"|ורשמי אלו המספרים על הרבוע והשווי יהיה כפי זה המשל ר"ל המצוייר בסוף זה השער והיא התמונה אשר עליה יהיו המרובעים השוים תמיד | ||
| + | |- | ||
| + | |It is clear from the illustrated example below that the sides of the square numbers in their succession increase according to the root of the production of the natural numbers: | ||
| + | |style="text-align:right;"|ומבואר נגלה מהדמיון המצוייר למטה שצלעות {{#annot:term|86,1263|jK4s}}המרובעים המספריים{{#annotend:jK4s}} כפי המשכם יתוספו כפי שרש צמיחת המספרים הטבעיים | ||
| + | |- | ||
| + | | | ||
| + | *The side of the first in potentia, which is 1, is 1. | ||
| + | |style="text-align:right;"|כי צלע הראשון בכח אשר הוא האחד אחד | ||
| + | |- | ||
| + | | | ||
| + | *The side of the first in actu is 2. | ||
| + | |style="text-align:right;"|וצלע הראשון בפעל ב‫' | ||
| + | |- | ||
| + | | | ||
| + | *The side of the second in actu is 3. | ||
| + | |style="text-align:right;"|וצלע השני בפעל ג‫' | ||
| + | |- | ||
| + | | | ||
| + | *The side of the third in actu is 4. | ||
| + | |style="text-align:right;"|וצלע השלישי בפעל ד‫' | ||
| + | |- | ||
| + | |And so is their production always. | ||
| + | |style="text-align:right;"|וכן צמיחתם תמיד | ||
| + | |- | ||
| + | |It is already said in the first chapter how the equilateral numbers are produced, as it was mentioned there. | ||
| + | |style="text-align:right;"|וכבר אמרנו במאמר הראשון איך יתילדו המספרים השוי הצלעות למה שקרה שם זכרו | ||
| + | |- | ||
| + | |Yet, it is necessary to return to discuss it, since this is the place of its particular mention. | ||
| + | |style="text-align:right;"|ואמנם מחוייב להשיב המאמר עליו הנה אחר שהוא מקום זכרו המיוחד בו | ||
| + | |- | ||
| + | |It is said that the production of the square numbers is from the successive addition of the natural odd numbers. | ||
| + | |style="text-align:right;"|ונאמר שצמיחת אלו המרובעים מ{{#annot:term|154,2511|SHEd}}הוספת{{#annotend:SHEd}} הנפרדים הטבעיים קצתם על קצת | ||
| + | |- | ||
| + | | | ||
| + | *Their beginning is the one, which is an odd in potentia and a square in potentia. | ||
| + | |style="text-align:right;"|אחר שהתחלתם האחד אשר הוא נפרד בכח ומרובע בכח | ||
| + | |- | ||
| + | | | ||
| + | *The three, which are the first odd, when added to the one, the result is four, which is the first actual square. <math>\scriptstyle{\color{blue}{1+3=4}}</math> | ||
| + | |style="text-align:right;"|כי השלשה אשר הם נפרד ראשון כאשר נוספו על האחד היה הגעת זה ארבעה והוא המרובע הראשון בפעל | ||
| + | |- | ||
| + | | | ||
| + | *When the second odd number, which is five, is added to the first actual square, the result is nine, which is the second actual square. <math>\scriptstyle{\color{blue}{4+5=9}}</math> | ||
| + | |style="text-align:right;"|וכאשר נוסף על המרובע הראשון בפעל הנפרד השני אשר הוא חמשה היה הגעת זה תשעה והוא המרובע השני בפעל | ||
| + | |- | ||
| + | |Since it was already mentioned once, there is no need to elaborate about it more than by saying that the production of the equilateral squares is by adding the natural odd numbers to each other. | ||
| + | |style="text-align:right;"|ולפי שאנו כבר זכרנו זה פעם אחת אין צורך לנו להאריך ביותר מאשר נאמר שהולדת המרובעים השוי הצלעות אמנם תהיה בתוספת הנפרדים הטבעיים קצתם על קצת | ||
| + | |- | ||
| + | |Each side of any square among them is a root of that square, i.e. when it is counted by the measure of the units that are in it, the result is that square. | ||
| + | |style="text-align:right;"|וכל צלע מצלעות כל מרובע מהם הוא שרש אותו המרובע ר"ל כאשר ימנה בשעורי מה שבו מהאחדים היה כללו הגעת אותו המרובע | ||
| + | |- | ||
| + | |When the roots of these squares are set successively, they accord with the order of the succession of the natural numbers. | ||
| + | |style="text-align:right;"|וכאשר הונחו שרשי אלו המרובעים ילוו קצתם לקצת היו {{#annot:term|2576,2575|pHYO}}על סדר המספרים הטבעיים ימשכו קצתם לקצת{{#annotend:pHYO}} | ||
| + | |- | ||
| + | |The rule of this discussion is in accordance with this. | ||
| + | |style="text-align:right;"|וכלל זה המאמר על זה | ||
| + | |- | ||
| + | |This is the diagram of the squares, which was intended to be brought at the end of the discussion: | ||
| + | |style="text-align:right;"|וזה צורת המרובעים אשר יעדנו להביאה בסוף הדברים | ||
| + | |- | ||
| + | | | ||
| + | |[[File:Square Numbers.png|thumb|150px|right]] | ||
| + | |- | ||
| + | | | ||
| + | *The first potential square is one, which is written in black, on the right upper angle, upon which א is written. | ||
| + | |style="text-align:right;"|המרובע הראשון בכח הוא האחד הנרשם בשחרות בזוית העליונה מצד הימין והוא הנרשם עליו א‫' | ||
| + | |- | ||
| + | | | ||
| + | *The first actual square is the sum of this one and the three red units that follow, by the property of the geometrical shape. | ||
| + | |style="text-align:right;"|והמרובע הראשון בפעל הוא המתקבץ מזה האחד ומשלשת האחדים האדומים אשר ילוו עליהם על תכונת תמונת הרושם | ||
| + | |- | ||
| + | | | ||
| + | *The second square is the sum of this first actual square and five units. | ||
| + | |style="text-align:right;"|והמרובע השני הוא המתקבץ מזה המרובע הראשון בפועל ומחמשת אחדים | ||
| + | |- | ||
| + | | | ||
| + | *The third actual square is the sum of this second actual square and the seven red units that follow, by the property of the geometrical shape. | ||
| + | |style="text-align:right;"|והמרובע השלישי בפעל הוא המתקבץ מזה המרובע השני בפעל ומשבעה האחדים האדומים הנלוים עליהם על תכונת תבנית הרושם | ||
| + | |- | ||
| + | | | ||
| + | *The fourth actual square is the sum of this third actual square and the nine black units that follow, by the property of the geometrical shape. | ||
| + | |style="text-align:right;"|והמרובע הרביעי בפעל הוא המתקבץ מזה המרובע השלישי בפעל ומתשעת האחדים השחורים הנלוים עליהם על תכונת תבנית הרושם | ||
| + | |- | ||
| + | |It should be examined that the geometrical shapes in this diagram are [the sums of] the natural odd numbers, according to their succession in the natural order. | ||
| + | |style="text-align:right;"|וממה שראוי לך שתבחנהו שתמונות הרושם בזאת הצורה הם הנפרדים הטבעיים כפי המשכם בסדר הטבע | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ====== The Discussion on the Pentagonal Numbers, their Production and their Sides ====== | ||
| + | |||
| + | |style="text-align:right;"|<big>הדבור במחומשים המספרים והתילדם וצלעותיהם</big> | ||
| + | |- | ||
| + | |The pentagonal number, i.e. all pentagonal numbers, their production is not through the production of the triangle and the square, since the pentagon has five sides and five angles, such as: 1, 5, 12, 22, 35, 51, and the descriptions of these numbers by the quintuple and equality are as drawn at the end of this chapter. | ||
| + | |style="text-align:right;"|ואמנם המספר המחומש ר"ל כלל המחומשים המספרים אין צמיחתם בצמיחת המשלש והמרובע כי המחומש לו חמשה צלעות וחמשה זויות כמו א' ה' י"ב כ"ב ל"ה נ"א ורשמי אלו המספרים על החמוש והשווי הם כפי מה שציירתי לך בסוף זה השער | ||
| + | |- | ||
| + | | | ||
| + | *The side of the first actual pentagon, which is 5, is 2. | ||
| + | |style="text-align:right;"|הנה צלע המחומש הראשון בפעל והוא ה' שנים | ||
| + | |- | ||
| + | | | ||
| + | *The side of the second actual pentagon, which is 12, is 3. | ||
| + | |style="text-align:right;"|וצלע המחומש השני בפעל והוא י"ב שלשה | ||
| + | |- | ||
| + | | | ||
| + | *The side of the third actual pentagon, which is 22, is 4. | ||
| + | |style="text-align:right;"|וצלע המחומש השלישי בפעל והוא כ"ב ארבעה | ||
| + | |- | ||
| + | |It is necessary that the sides of these pentagons follow each other as the sequence of the natural numbers, as clarified concerning the triangular and the square numbers. | ||
| + | |style="text-align:right;"|וכן יחוייב שיהיו צלעות אלו המחומשים נמשכים יחד {{#annot:term|2576,2575|mtpu}}על סדר המספרים הטבעיים{{#annotend:mtpu}} כפי מה שהתבאר במשולש והמרובע | ||
| + | |- | ||
| + | |The production of the pentagonal numbers: | ||
| + | |style="text-align:right;"|ואמנם התילד המחומש | ||
| + | |- | ||
| + | | | ||
| + | *It is already clarified that, since the first actual pentagon is 5, the second is 12, and the third is 22, what is added to the first potential pentagon, which is 1, until it becomes the first actual pentagon, is 4. | ||
| + | |style="text-align:right;"|הנה כבר התבאר כי אחר שהיה המחומש הראשון בפעל ה' והשני י"ב והשלישי כ"ב שאשר הוספנו אותו על המחומש הראשון אשר הוא א' בכח ארבעה עד שהיה המחומש הראשון בפעל | ||
| + | |- | ||
| + | | | ||
| + | *When 7 is added to the first actual, which is 5, so that it becomes 12, which is the second actual, the excess of the 7, which was added at the end, over the 4, which was added first, is 3. | ||
| + | |style="text-align:right;"|וכאשר הוספנוהו על הראשון בפעל והוא ה' ז' עד שהיה י"ב והוא השני בפעל הנה מותר השבעה אשר הוספנום לסוף על הארבעה אשר הוספנום ראשונה שלשה | ||
| + | |- | ||
| + | | | ||
| + | :The second actual pentagonal number is 12, and the third actual is 22. | ||
| + | |style="text-align:right;"|והמחומש השני בפעל י"ב והשלישי בפועל כ"ב | ||
| + | |- | ||
| + | | | ||
| + | *What is added to the second actual, so that it becomes the third actual, is 10, and 10 exceeds 7 by 3. | ||
| + | |style="text-align:right;"|ואשר הוספנוהו על השני בפועל עד שיהיה השלישי בפעל עשרה ועשרה יותר משבעה שלשה | ||
| + | |- | ||
| + | |Hence, it is clear that the production of the pentagonal numbers is always by adding three to what was added to the preceding pentagonal number, and so it will be found always. | ||
| + | |style="text-align:right;"|הנה אם כן כבר התבאר שתולדת המחומשים בתוספת שלשה לעולם על מה שהוספנוהו במחומש אשר לפניו וכן ימצא תמיד | ||
| + | |- | ||
| + | | | ||
| + | *The fourth pentagonal number is 35, and 35 exceeds 22 by the addition of 13, and 13 exceeds 10 by 3. | ||
| + | |style="text-align:right;"|כי הנה המחומש הרביעי ל"ה ול"ה יותר מכ"ב בתוספת י"ג וי"ג יותר מי' שלשה | ||
| + | |- | ||
| + | |Thus, it is clear how the pentagonal numbers are produced. | ||
| + | |style="text-align:right;"|הנה אם כן כבר התבאר איך יתילדו המחומשים | ||
| + | |- | ||
| + | |Furthermore, it will be clear from all that is said, how the hexagonal and the heptagonal numbers and the others are composed: | ||
| + | |style="text-align:right;"|וגם כן כבר יתבאר מכל מה שאמרנו איך יתרכבו כמו כן המשושים והמשובעים וזולתם | ||
| + | |- | ||
| + | | | ||
| + | *It was explained that the triangular numbers are generated from the successive addition of the natural numbers, and the natural numbers are added one by one. | ||
| + | |style="text-align:right;"|וזה שאנו כבר בארנו שהמשלשים יתחדשו בתוספת המספרים הטבעיים קצתם על קצת והמספרים הטבעיים יתוספו באחד אחד | ||
| + | |- | ||
| + | | | ||
| + | *It was also explained that the square numbers are generated from the successive addition of the odd numbers, and the odd numbers are added two by two. | ||
| + | |style="text-align:right;"|ובארנו גם כן כי המרובעים יתחדשו בתוספת המספרים הנפרדים קצתם על קצת והנפרדים יתוספו שנים שנים | ||
| + | |- | ||
| + | | | ||
| + | *It was explained that the pentagonal numbers are generated from the addition of the numbers that are added successively three by three. | ||
| + | |style="text-align:right;"|ובארנו שהמחומשים יתחדשו בתוספת המספרים אשר יתוספו קצתם על קצת שלשה שלשה | ||
| + | |- | ||
| + | | | ||
| + | *It is said that the hexagonal numbers are generated from the addition of the numbers that are added successively four by four. | ||
| + | |style="text-align:right;"|ונאמר שהמשושים יתחדשו בתוספת המספרים אשר יתוספו קצתם על קצת ארבעה ארבעה | ||
| + | |- | ||
| + | | | ||
| + | *The heptagonal numbers are generated from the addition of the numbers that are added successively five by five. | ||
| + | |style="text-align:right;"|והמשובעים יתחדשו בהוספת המספרים אשר יתוספו קצתם על קצת חמשה חמשה | ||
| + | |- | ||
| + | |So also for the octagonal and the nonagonal numbers, or whatever one wishes of these, they are generated as this drawn description. | ||
| + | |style="text-align:right;"|וכן המשומין והמתושע או מה שתרצה מזה אמנם יתחדש בזה התאר המצוייר | ||
| + | |- | ||
| + | |Examples were already given for the pentagonal, hexagonal, heptagonal, and octagonal numbers. | ||
| + | |style="text-align:right;"|הנה כבר המשלנו דמיונים למחומשים ולמשושים ולמשובעים ולמשומנים | ||
| + | |- | ||
| + | | | ||
| + | :The numbers by which each plane number among them exceeds its preceding are distinguished by two colors. | ||
| + | |style="text-align:right;"|והבדלנו בחלוק שני גוונים המספרים אשר בם יוסיף כל מספר משוטח מהם על אשר לפניו | ||
| + | |- | ||
| + | |These numbers were assumed as an image of geometrical shapes, in order that what was mentioned will be clear, that the shapes of each species of the plane equi-angular numbers are produced from the addition of numbers that exceed [one another] by one number that never changes forever. | ||
| + | |style="text-align:right;"|והנחנו אותם המספרים כדמות תבניות הרושם כדי שיתבאר בם מה שזכרנו מאשר כל מין ממיני המספרים המשוטחים בעלי הזויות השוות אמנם יתילדו תמונותיו בתוספת מספרים יעדיפו במספר אחד לא ישתנה לעולם אל בלתי תכלית | ||
| + | |- | ||
| + | | | ||
| + | *The squares - the numbers that are added in them, i.e. the numbers of the shapes that complete them at the ends of the geometrical shapes exceed each other two by two. | ||
| + | |style="text-align:right;"|אמנם המרובעים המספרים אשר יתוספו בם ר"ל מספרי התמונות אשר ישלימו אותם על גבולי תמונות הרושם תהיה העדפתם שנים שנים | ||
| + | |- | ||
| + | | | ||
| + | *The pentagons - the excess mentioned with regard to them is three by three. | ||
| + | |style="text-align:right;"|ואמנם המחומשים הנה העודף הנזכר בם יהיה שלשה שלשה | ||
| + | |- | ||
| + | |Likewise, for any rank shifted forward in the ranks of the species of the plane numbers, the excess mentioned exceeds by one. | ||
| + | |style="text-align:right;"|וכמו כן כל מה שתעתיק מדרגה אחת במדרגות מיני המספרים המשוטחים יוסיף היתרון הנזכר באחד | ||
| + | |} | ||
| + | |||
| + | {|class="wikitable" style="margin-left: auto; margin-right: auto; text-align:center;" | ||
| + | |- | ||
| + | |משולש ח||משולש ז||משולש ו||משולש ה||משולש ד||משולש ג||משולש ב||משולש א | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto;" | ||
| + | |- | ||
| + | |colspan="3"|לו | ||
| + | |- | ||
| + | |כח||ז||א | ||
|} | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto;" | ||
| + | |- | ||
| + | |colspan="3"|כח | ||
| + | |- | ||
| + | |כא||ו||א | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto;" | ||
| + | |- | ||
| + | |colspan="3"|כא | ||
| + | |- | ||
| + | |טו||ה||א | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto;" | ||
| + | |- | ||
| + | |colspan="3"|טו | ||
| + | |- | ||
| + | |י||ד||א | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto;" | ||
| + | |- | ||
| + | |colspan="3"|י | ||
| + | |- | ||
| + | |ו||ג||א | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto;" | ||
| + | |- | ||
| + | |colspan="3"|ו | ||
| + | |- | ||
| + | |ג||ב||א | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto;" | ||
| + | |- | ||
| + | |colspan="2"|ג | ||
| + | |- | ||
| + | |ב||א | ||
| + | |} | ||
| + | |א | ||
| + | |- | ||
| + | |מרובע ח||מרובע ז||מרובע ו||מרובע ה||מרובע ד||מרובע ג||מרובע ב||מרובע א | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto;" | ||
| + | |- | ||
| + | |colspan="3"|סד | ||
| + | |- | ||
| + | |מט||יג||ב | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto;" | ||
| + | |- | ||
| + | |colspan="3"|מט | ||
| + | |- | ||
| + | |לו||יא||ב | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto;" | ||
| + | |- | ||
| + | |colspan="3"|לו | ||
| + | |- | ||
| + | |כה||ט||ב | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto;" | ||
| + | |- | ||
| + | |colspan="3"|כה | ||
| + | |- | ||
| + | |יו||ז||ב | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto;" | ||
| + | |- | ||
| + | |colspan="3"|יו | ||
| + | |- | ||
| + | |ט||ה||ב | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto;" | ||
| + | |- | ||
| + | |colspan="3"|ט | ||
| + | |- | ||
| + | |ד||ג||ב | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto;" | ||
| + | |- | ||
| + | |colspan="2"|ד | ||
| + | |- | ||
| + | |ב||ב | ||
| + | |} | ||
| + | |א | ||
| + | |- | ||
| + | |מחומש ח||מחומש ז||מחומש ו||מחומש ה||מחומש ד||מחומש ג||מחומש ב||מחומש א | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto;" | ||
| + | |- | ||
| + | |colspan="3"|צב | ||
| + | |- | ||
| + | |ע||יט||ג | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto;" | ||
| + | |- | ||
| + | |colspan="3"|ע | ||
| + | |- | ||
| + | |נא||יו||ג | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto;" | ||
| + | |- | ||
| + | |colspan="3"|נא | ||
| + | |- | ||
| + | |לה||יג||ג | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto;" | ||
| + | |- | ||
| + | |colspan="3"|לה | ||
| + | |- | ||
| + | |כב||י||ג | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto;" | ||
| + | |- | ||
| + | |colspan="3"|כב | ||
| + | |- | ||
| + | |יב||ז||ג | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto;" | ||
| + | |- | ||
| + | |colspan="3"|יב | ||
| + | |- | ||
| + | |ה||ד||ג | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto;" | ||
| + | |- | ||
| + | |colspan="2"|ה | ||
| + | |- | ||
| + | |ב||ג | ||
| + | |} | ||
| + | |א | ||
| + | |- | ||
| + | |משושה ח||משושה ז||משושה ו||משושה ה||משושה ד||משושה ג||משושה ב||משושה א | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto;" | ||
| + | |- | ||
| + | |colspan="3"|קכ | ||
| + | |- | ||
| + | |צא||כה||ד | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto;" | ||
| + | |- | ||
| + | |colspan="3"|צא | ||
| + | |- | ||
| + | |סו||כא||ד | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto;" | ||
| + | |- | ||
| + | |colspan="3"|סו | ||
| + | |- | ||
| + | |מה||יז||ד | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto;" | ||
| + | |- | ||
| + | |colspan="3"|מה | ||
| + | |- | ||
| + | |כח||יג||ד | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto;" | ||
| + | |- | ||
| + | |colspan="3"|כח | ||
| + | |- | ||
| + | |טו||ט||ד | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto;" | ||
| + | |- | ||
| + | |colspan="3"|טו | ||
| + | |- | ||
| + | |ו||ה||ד | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto;" | ||
| + | |- | ||
| + | |colspan="2"|ו | ||
| + | |- | ||
| + | |ב||ד | ||
| + | |} | ||
| + | |א | ||
| + | |} | ||
| + | |||
| + | {|class="wikitable" style="color:blue; margin-left: auto; margin-right: auto; text-align:center;" | ||
| + | |- | ||
| + | |triangle 8||triangle 7||triangle 6||triangle 5||triangle 4||triangle 3||triangle 2||triangle 1 | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto;" | ||
| + | |- | ||
| + | |colspan="3"|36 | ||
| + | |- | ||
| + | |28||7||1 | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto;" | ||
| + | |- | ||
| + | |colspan="3"|28 | ||
| + | |- | ||
| + | |21||6||1 | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto;" | ||
| + | |- | ||
| + | |colspan="3"|21 | ||
| + | |- | ||
| + | |15||5||1 | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto;" | ||
| + | |- | ||
| + | |colspan="3"|15 | ||
| + | |- | ||
| + | |10||4||1 | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto;" | ||
| + | |- | ||
| + | |colspan="3"|10 | ||
| + | |- | ||
| + | |6||3||1 | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto;" | ||
| + | |- | ||
| + | |colspan="3"|6 | ||
| + | |- | ||
| + | |3||2||1 | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto;" | ||
| + | |- | ||
| + | |colspan="2"|3 | ||
| + | |- | ||
| + | |2||1 | ||
| + | |} | ||
| + | |1 | ||
| + | |- | ||
| + | |square 8||square 7||square 6||square 5||square 4||square 3||square 2||square 1 | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto;" | ||
| + | |- | ||
| + | |colspan="3"|64 | ||
| + | |- | ||
| + | |49||13||2 | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto;" | ||
| + | |- | ||
| + | |colspan="3"|49 | ||
| + | |- | ||
| + | |36||11||2 | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto;" | ||
| + | |- | ||
| + | |colspan="3"|36 | ||
| + | |- | ||
| + | |25||9||2 | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto;" | ||
| + | |- | ||
| + | |colspan="3"|25 | ||
| + | |- | ||
| + | |16||7||2 | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto;" | ||
| + | |- | ||
| + | |colspan="3"|16 | ||
| + | |- | ||
| + | |9||5||2 | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto;" | ||
| + | |- | ||
| + | |colspan="3"|9 | ||
| + | |- | ||
| + | |4||3||2 | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto;" | ||
| + | |- | ||
| + | |colspan="2"|4 | ||
| + | |- | ||
| + | |2||2 | ||
| + | |} | ||
| + | |1 | ||
| + | |- | ||
| + | |pentagon 8||pentagon 7||pentagon 6||pentagon 5||pentagon 4||pentagon 3||pentagon 2||pentagon 1 | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto;" | ||
| + | |- | ||
| + | |colspan="3"|92 | ||
| + | |- | ||
| + | |70||19||3 | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto;" | ||
| + | |- | ||
| + | |colspan="3"|70 | ||
| + | |- | ||
| + | |51||16||3 | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto;" | ||
| + | |- | ||
| + | |colspan="3"|51 | ||
| + | |- | ||
| + | |35||13||3 | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto;" | ||
| + | |- | ||
| + | |colspan="3"|35 | ||
| + | |- | ||
| + | |22||10||3 | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto;" | ||
| + | |- | ||
| + | |colspan="3"|22 | ||
| + | |- | ||
| + | |12||7||3 | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto;" | ||
| + | |- | ||
| + | |colspan="3"|12 | ||
| + | |- | ||
| + | |5||4||3 | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto;" | ||
| + | |- | ||
| + | |colspan="2"|5 | ||
| + | |- | ||
| + | |2||3 | ||
| + | |} | ||
| + | |1 | ||
| + | |- | ||
| + | |hexagon 8||hexagon 7||hexagon 6||hexagon 5||hexagon 4||hexagon 3||hexagon 2||hexagon 1 | ||
| + | |- | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto;" | ||
| + | |- | ||
| + | |colspan="3"|120 | ||
| + | |- | ||
| + | |91||25||4 | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto;" | ||
| + | |- | ||
| + | |colspan="3"|91 | ||
| + | |- | ||
| + | |66||21||4 | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto;" | ||
| + | |- | ||
| + | |colspan="3"|66 | ||
| + | |- | ||
| + | |45||17||4 | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto;" | ||
| + | |- | ||
| + | |colspan="3"|45 | ||
| + | |- | ||
| + | |28||13||4 | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto;" | ||
| + | |- | ||
| + | |colspan="3"|28 | ||
| + | |- | ||
| + | |15||9||4 | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto;" | ||
| + | |- | ||
| + | |colspan="3"|15 | ||
| + | |- | ||
| + | |6||5||4 | ||
| + | |} | ||
| + | | | ||
| + | {|style="margin-left: auto; margin-right: auto;" | ||
| + | |- | ||
| + | |colspan="2"|6 | ||
| + | |- | ||
| + | |2||4 | ||
| + | |} | ||
| + | |1 | ||
| + | |} | ||
| + | |||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ====== The Discussion about how All Plane Polygonals Consist of and are Resolved into Triangles ====== | ||
| + | |||
| + | |style="text-align:right;"|<big>הדבור באשר כל המשוטחים בעלי הזויות השוות מהמשולשים יתרכבו ואליהם יותכו</big> | ||
| + | |- | ||
| + | |The explanation of what was said before, that the polygonal numbers are composed of the triangles and are resolved into them, follows from this. | ||
| + | |style="text-align:right;"|וכבר קרה מזה ביאור מה שאמרנו לפנים ש{{#annot:term|88,2568|LmEf}}בעלי המספרים הישרי הקוים השוי הזויות{{#annotend:LmEf}} הם מורכבים מהמשולשים ונתכים אליהם | ||
| + | |- | ||
| + | | | ||
| + | *It is evident that the squares are produced from the composition of the triangles: | ||
| + | |style="text-align:right;"|וזה שכבר נראה לנו שהמרובעים אמנם חודשו מהרכבת המשולשים | ||
| + | |- | ||
| + | | | ||
| + | :*Setting the triangles successively, as 1; 3; 6; 10; 15 | ||
| + | |style="text-align:right;"|כאשר הנחנו המשולשים על סדר כמו א' ג' ו' י' ט"ו | ||
| + | |- | ||
| + | | | ||
| + | ::*When the first potential triangle is added to the first actual triangle, which is 3, the first actual square is created, which is 4. | ||
| + | :::<math>\scriptstyle{\color{blue}{1+3=4}}</math> | ||
| + | |style="text-align:right;"|עוד חברנו המשלש הראשון בכח אל המשלש הראשון בפעל והוא ג' יחודש המרובע הראשון בפעל והוא ד‫' | ||
| + | |- | ||
| + | | | ||
| + | ::*When the first actual triangle, which is 3, is added to the second actual triangle, which is 6, the second actual square is created, which is 9. | ||
| + | :::<math>\scriptstyle{\color{blue}{3+6=9}}</math> | ||
| + | |style="text-align:right;"|וכאשר חברנו המשולש הראשון בפעל אשר הוא ג' אל השני בפעל אשר הוא ו' יתחדש המרובע השני בפעל אשר הוא ט‫' | ||
| + | |- | ||
| + | | | ||
| + | ::*When the second actual triangle, which is 6, is added to the third actual triangle, which is 10, the third actual square is created, which is 16. | ||
| + | :::<math>\scriptstyle{\color{blue}{6+10=16}}</math> | ||
| + | |style="text-align:right;"|וכן כאשר חברנו המשולש השני בפעל והוא ו' אל המשולש השלישי בפועל והוא י' יחודש המרובע השלישי והוא י"ו | ||
| + | |- | ||
| + | | | ||
| + | :You will find it always so, from every two triangles a square is composed by the order that was demonstrated. | ||
| + | |style="text-align:right;"|וכן תמצא זה תמיד מתרכב מכל שני משולשים מהם מרובע כפי זה הסדר אשר המשלנו | ||
| + | |- | ||
| + | | | ||
| + | :Also in a reverse statement: every square is resolved into the triangles from which it is composed. | ||
| + | |style="text-align:right;"|ובהפך זה המאמר יותך כל מרובע מהמרובעים אל המשולשים אשר מהם הורכב | ||
| + | |- | ||
| + | | | ||
| + | :*4, which is a square, is resolved into 1 and 3, which are two triangles. | ||
| + | |style="text-align:right;"|כי הארבעה אשר הוא מרובע יותך אל אחד ושלשה והם שני משולשים | ||
| + | |- | ||
| + | | | ||
| + | :*9 into 3 and 6, which are two triangles. | ||
| + | |style="text-align:right;"|והתשעה אל שלשה וששה הם שני משולשים | ||
| + | |- | ||
| + | | | ||
| + | :Thus, it is clear how the squares are composed of the triangles and resolved into them. | ||
| + | |style="text-align:right;"|הנה כבר התבאר איך יתרכבו המרובעים מן המשולשים ויתכו אליהם | ||
| + | |- | ||
| + | | | ||
| + | *The pentagons, as will be described, are composed of the triangles and the squares: | ||
| + | |style="text-align:right;"|ואמנם המחומשים כפי מה שאתאר וזה שהם יתרכבו מהמשולשים והמרובעים | ||
| + | |- | ||
| + | | | ||
| + | :*Arranging the triangles in a line, as 1; 3; 6; 10; 15 | ||
| + | |style="text-align:right;"|כאשר סדרנו המשולשים בטור כמו א' ג' ו' י' ט"ו | ||
| + | |- | ||
| + | | | ||
| + | :*Setting the squares in a line, as 4; 9; 16; 25; 36 | ||
| + | |style="text-align:right;"|עוד נסדר המרובעים בטור כמו ד' ט' י"ו כ"ה ל"ו | ||
| + | |- | ||
| + | | | ||
| + | :*Summing each term with its counterpart. | ||
| + | |style="text-align:right;"|עוד נחבר כל מדרגה עם נכחה | ||
| + | |- | ||
| + | | | ||
| + | ::*The result of the sum of the first potential triangle, which is 1, with the first actual square, which is 4, is the first actual pentagon, which is 5. | ||
| + | :::<math>\scriptstyle{\color{blue}{1+4=5}}</math> | ||
| + | |style="text-align:right;"|הנה יתקבץ מחבור המשלש הראשון בכח אשר הוא א' עם המרובע הראשון בפעל אשר הוא ד' המחומש הראשון בפעל אשר הוא ה‫' | ||
| + | |- | ||
| + | | | ||
| + | :You will find it always so, when you will compose it according to this order. | ||
| + | |style="text-align:right;"|וכן תמצאהו תמיד כאשר תרכיבהו כפי זה הסדר | ||
| + | |- | ||
| + | | | ||
| + | :In the reverse statement: it is clear that pentagons are resolved into the triangles and into what is composed of the triangles, i.e. the squares. | ||
| + | |style="text-align:right;"|ובהפך זה המאמר יתבאר שהמחומשים יותכו אל המשולשים ואל מה שיורכב מהמשולשים ר"ל המרובעים | ||
| + | |- | ||
| + | | | ||
| + | :*5 is resolved into 1 and 4. | ||
| + | |style="text-align:right;"|כי החמשה יותך אל אחד וארבעה | ||
| + | |- | ||
| + | | | ||
| + | :*12 into 3 and 9. | ||
| + | |style="text-align:right;"|והי"ב אל שלשה ותשעה | ||
| + | |- | ||
| + | | | ||
| + | :*And so on for what follows. | ||
| + | |style="text-align:right;"|וכן מה שאחר זה | ||
| + | |- | ||
| + | | | ||
| + | :Thus, it is clear how the pentagons are composed of the triangles and are resolved into them. | ||
| + | |style="text-align:right;"|הנה כבר התבאר איך יתרכבו המחומשים מהמשולשים ויותכו אליהם | ||
| + | |- | ||
| + | | | ||
| + | *Likewise, you will find that the hexagons are also produced from the composition of the triangles with the their counterparts in the line of the pentagons, when they are composed similarly to what was explained. | ||
| + | |style="text-align:right;"|וכן תמצא גם כן שיתילדו המשושים מהרכבת המשולשים עם מה שיהיה נכחם בטור המחומשים כאשר הורכבו על דמיון מה שבארנו | ||
| + | |- | ||
| + | | | ||
| + | :Except that the beginning of the triangles that are set is the first potential triangle, which is one, while the first term of the numbers of the other surfaces is their first actual term. | ||
| + | |style="text-align:right;"|אלא שיהיה לעולם ראש הנחת המשולשים המשולש הראשון בכח אשר הוא אחד וראש מדרגות מספרי השטחים האחרים הראשון מהם בפעל | ||
| + | |- | ||
| + | | | ||
| + | :*The first actual hexagon is created from the combination of the first potential triangle, which is 1, with the first actual pentagon, which is 5. | ||
| + | ::<math>\scriptstyle{\color{blue}{1+5=6}}</math> | ||
| + | |style="text-align:right;"|והמשושה הראשון בפעל אמנם יחודש מהרכבת המשלש הראשון בכח אשר הוא האחד עם המחומש הראשון בפעל אשר הוא חמשה | ||
| + | |- | ||
| + | | | ||
| + | :*The second hexagon [is created] from the combination of the first actual triangle, which is 3, with the second actual pentagon, which is 12. | ||
| + | ::<math>\scriptstyle{\color{blue}{3+12=15}}</math> | ||
| + | |style="text-align:right;"|והמשושה השני מהרכבת המשלש הראשון בפעל אשר הוא ג' עם המחומש השני בפעל אשר הוא י"ב | ||
| + | |- | ||
| + | | | ||
| + | :*The first actual heptagon is created from the combination of the first potential triangle, which is 1, with the first actual hexagon. | ||
| + | ::<math>\scriptstyle{\color{blue}{1+6=7}}</math> | ||
| + | |style="text-align:right;"|וכן המשובע גם כן יתחדש הראשון ממנו בפעל מהרכבת המשלש הראשון בכח אשר הוא א' עם המשושה הראשון בפעל | ||
| + | |- | ||
| + | | | ||
| + | :*The second heptagon [is created] from the combination of the first actual triangle, with the second actual hexagon. | ||
| + | ::<math>\scriptstyle{\color{blue}{3+15=18}}</math> | ||
| + | |style="text-align:right;"|והמשובע השני בפעל מהרכבת המשולש הראשון בפעל עם המשושה השני בפעל | ||
| + | |- | ||
| + | |So, all of them - the octagons, the nonagons and the other polygons - are always found, their production is from the triangles and they resolve into the triangle. | ||
| + | |style="text-align:right;"|וכן ימצאו כלם תמיד המשומנים והמתושעים וזולתם מבעלי הזויות ותולדותם מן המשולשים והתכתם אל המשולש | ||
| + | |- | ||
| + | |To explain it to the sense [i.e. visually] we set a diagram of parallel lines [i.e. table]: the first line to the triangles; the second to the squares; the third to the pentagons; the fourth to the hexagons; the fifth line to the heptagons; and the sixth line to the octagons. | ||
| + | |style="text-align:right;"|ולבאר זה לחוש נניח לו צורה מטורים נכחים הטור הראשון למשולשים והשני למרובעים והשלישי למחומשים והרביעי למשושים והטור החמישי למשובעים והטור הששי למשומנים | ||
| + | |- | ||
| + | |Whoever wants to add numbers of surfaces to this diagram should stick to the order we relied on and aimed at. | ||
| + | |style="text-align:right;"|ומי שירצה להוסיף בזאת הצורה מספרים מבעלי השטחים ידבק בסדור אשר נשעננו וכוננו בו אליו | ||
| + | |- | ||
| + | |What is clear from this diagram is that: | ||
| + | |style="text-align:right;"|וממה שיתבאר בזאת הצורה | ||
| + | |} | ||
| + | |||
| + | {|class="wikitable" style="margin-left: auto; margin-right: auto; text-align:center;" | ||
| + | |- | ||
| + | |י||ט||ח||ז||ו||ה||ד||ג||ב||א||מספר הצלעות||מספר השטחים||א | ||
| + | |- | ||
| + | |נה||מה||לו||כח||כא||טו||י||ו||ג||א||המשולשים||א||ב | ||
| + | |- | ||
| + | |ק||פא||סד||מט||לו||כה||יו||ט||ד||א||המרובעים||ב||ג | ||
| + | |- | ||
| + | |קמה||קיז||צב||ע||נא||לה||כב||יב||ה||א||המחומשים||ג||ד | ||
| + | |- | ||
| + | |קצ||קנג||קכ||צא||סו||מה||כח||טו||ו||א||המשושים||ד||ה | ||
| + | |- | ||
| + | |רלה||קפט||קמח||קיב||פא||נה||לד||יח||ז||א||המשובעים||ה||ו | ||
| + | |- | ||
| + | |רפ||רכה||קעו||קלג||צו||סה||מ||כא||ח||א||המשומנים||ו||ז | ||
| + | |} | ||
| + | {|class="wikitable" style="color: blue; margin-left: auto; margin-right: auto; text-align:center;" | ||
| + | |- | ||
| + | |10||9||8||7||6||5||4||3||2||1||no. of sides||surface no.||1 | ||
| + | |- | ||
| + | |55||45||36||28||21||15||10||6||3||1||triangles||1||2 | ||
| + | |- | ||
| + | |100||81||64||49||36||25||16||9||4||1||squares||2||3 | ||
| + | |- | ||
| + | |145||117||92||70||51||35||22||12||5||1||pentagonals||3||4 | ||
| + | |- | ||
| + | |190||153||120||91||66||45||28||15||6||1||hexagonals||4||5 | ||
| + | |- | ||
| + | |235||189||148||112||81||55||34||18||7||1||heptagonals||5||6 | ||
| + | |- | ||
| + | |280||225||176||133||96||65||40||21||8||1||octagonals||6||7 | ||
| + | |} | ||
| + | |||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | :*The first actual species, i.e. the numerical surfaces, exceed each other's breadth of shape [i.e. number] by the first potential triangle, which is one. | ||
| + | |style="text-align:right;"|שהמינים הראשונים בפעל ר"ל {{#annot:term|83,1568|xboU}}המשוטחים המספריים{{#annotend:xboU}} יעדיפו על המשכם ברחב הצורה במשלש הראשון בכח והוא האחד | ||
| + | |- | ||
| + | | | ||
| + | :*The second actual species exceed each other's breadth of shape [i.e. number] by the first actual triangle, i.e. by three. | ||
| + | |style="text-align:right;"|והמינים השניים בפעל יעדיפו על המשכם ברחב הצורה במשולש הראשון בפעל ר"ל בשלשה | ||
| + | |- | ||
| + | | | ||
| + | :*The third actual species exceed each other's breadth of shape [i.e. number] by the second actual triangle. | ||
| + | |style="text-align:right;"|והמינים השלישים בפעל יעדיפו על המשכם ברחב הצורה במשולש השני בפעל | ||
| + | |- | ||
| + | | | ||
| + | :*The fourth actual species exceed each other by the third actual triangle. | ||
| + | |style="text-align:right;"|וכן המינים הרביעים בפעל יעדיפו על המשכם במשלש השלישי בפעל | ||
| + | |- | ||
| + | | | ||
| + | :*And so on for what follows. | ||
| + | |style="text-align:right;"|וכן מה שאחר זה | ||
| + | |- | ||
| + | |The discussion on the plane polygonal numbers is complete. | ||
| + | |style="text-align:right;"|וכבר נשלם המאמר במספרים המשוטחים בעלי הזויות | ||
| + | |- | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ===== The Discussion on the Solid Numbers ===== | ||
| + | |||
| + | |style="text-align:right;"|<big>הדבור ב{{#annot:term|89,2571|mazQ}}מספרים הגרמיים{{#annotend:mazQ}}</big> | ||
| + | |- | ||
| + | |The distinction between the solid and the surface has already been explained in the previous discussion | ||
| + | |style="text-align:right;"|כבר בארנו במה שקדם מן המאמר מה ההפרש בין {{#annot:term|587,2570|rQJK}}הגרם{{#annotend:rQJK}} והשטח | ||
| + | |- | ||
| + | |{{#annot:definition|587,2570|kMEJ}}It was said that the solid adds a dimension of depth to the surface, since the surface has only length and breadth | ||
| + | |style="text-align:right;"|ואמרנו שהגרם הוא אשר יוסיף משך בעומק על השטח אחר שהשטח אורך ורחב לבד{{#annotend:kMEJ}} | ||
| + | |- | ||
| + | | | ||
| + | :Saying depth or height is the same. | ||
| + | |style="text-align:right;"|ואמרנו עומק או גובה הכל שוה | ||
| + | |- | ||
| + | |People have said it all before. | ||
| + | |style="text-align:right;"|וכבר אמרו כל זה אנשים | ||
| + | |- | ||
| + | |Since the discussion on triangles preceded in the section on surfaces, as it was more worthy of precedence due to the reason we indicated: that they are a principle and a root for all surfaces, the pyramids precede in the discussion on solid numbers. | ||
| + | |style="text-align:right;"|ואחר שהיה קודם במאמרנו על השטח הדבור על משולשים לעלה אשר תארנו מהיותר ראוי לקדימה אחר שהם פנה לכל השטחים ושרש יהיה הקדימנו המאמר הזה ב{{#annot:term|587,2570|Ff7g}}גרמים{{#annotend:Ff7g}} על המזונבים | ||
| + | |- | ||
| + | |By pyramids I mean those that begin in a rectilinear plane base, rising in height, until they culminate in one. | ||
| + | |style="text-align:right;"|ורצוני ב{{#annot:term|1097,1673|gLyL}}מזונבים{{#annotend:gLyL}} אשר יתחילו מתושבת משוטח ישר הקוים ר"ל הצלעות עוד יעלו בגובה עד שיכלו אל האחד | ||
| + | |- | ||
| + | |All the surfaces of this shape are triangles except its base, since the bases of the pyramid species are either triangles, or squares, or pentagons, or hexagons, or others of the mentioned surfaces, but its [other] surfaces are triangles only. | ||
| + | |style="text-align:right;"|כי זאת התמונה אם שיהיו שטחיה כלם משלשים מלבד תושבתה כי התושבות לזה המין המזונב כבר יהיו משולשים או מרובעים או מחומשים או משושים או זולת זה מהשטחים אשר זכרנו ואמנם בשטחיו כאשר היה {{#annot:term|1097,1673|Ofm0}}מזונב{{#annotend:Ofm0}} הם משולשים לבד | ||
| + | |- | ||
| + | |Since the pyramid whose bases are all triangles, i.e. the triangular pyramid is a principle and a root for all the pyramids, as they are composed from it and dissolve into it, it is necessary to begin with its description. | ||
| + | |style="text-align:right;"|ומפני שהמזונב אשר תושבותיו יחד משולשים ר"ל {{#annot:triangular|1097,1527|Gr1Y}}הגרם המשולש{{#annotend:Gr1Y}} פנה ושרש לכל הגרמים בעלי הזויות אחר שהרכבתם ממנו והתכתם אליו יחוייב שנתחיל בו לתארו | ||
| + | |- | ||
| + | |This solid is encompassed by four triangles. | ||
| + | |style="text-align:right;"|ונאמר שזה הגרם יקיפו בו ארבעה משולשים | ||
| + | |- | ||
| + | | | ||
| + | *If one imagines solid shapes from the two-dimensional shapes: an equilateral triangle of three dimensions that are lengthwise equal to its sides, going from the angles of its base, converging to one point, opposite to the center on the base of the height, then a pyramid is conceived, having four equal surfaces, one of them is the base, and the remaining three are encompassing it. | ||
| + | |style="text-align:right;"|כי אלו ידמה מדמה מהתמונות הגרמיות מהמדתיות משולש שוה הצלעות בעל שלשה המשכים שוים באורך לצלעותיו יצאו מזויות תושבתו עוד יפגשו על נקדה נכחית למרכז בנפילת עמוד יהיה כבר ידומה {{#annot:term|1097,2442|o7LK}}גרם מזדנב{{#annotend:o7LK}} לו ארבעה שטחים שוים אחד מהם התושבת והשלשה הנשארים המקיפים בו | ||
| + | |- | ||
| + | | | ||
| + | *If one imagines a square, four sides are going from its angles, one from each side of its base, and all converging at one point, opposite to the center in the falling of the base of height of the square, then a pyramid is conceived, i.e. a pyramid that has five surfaces, as this figure, one of which is a square surface, which is its base, and the remaining four are triangles that encompassing it. | ||
| + | |style="text-align:right;"|וכמו כן גם כן אלו ידמה מדמה מרובע שוה יצאו מזויותיו ארבע צלעות כל אחד מהם לצלע מצלעות תושבתו ויפגשו כלם על נקדה נכחית למרכז בנפילת עמוד המרובע ידומה גרם המזדנב ר"ל מחודד לו חמשה שטחים כמו זאת הצורה אחד מהם שטח מרובע והוא תושבתו והארבעה הנשארים משולשים והם המקיפים בו | ||
| + | |- | ||
| + | | | ||
| + | *Likewise if one imagines the same for a pentagon, or a hexagon, or a heptagon, or other than these, such that lines are going from their angles, converging at one point, opposite to their center, triangles will be generated from the lines that are going from the angles and the ends of their base, and it will be a pyramid. | ||
| + | |style="text-align:right;"|וגם כן אלו ידמה מדמה כמו זה במחומש והמשושה והמשובע וזולתם ימצא בכל אחד מהם המשכים יצאו מזויותיהם ויפגשו על נקדה לנגד מרכזיהם יחדשו באותם הקוים היוצאים מהזויות ותכליות תושבתם משולשים ויהיה הגרם מזדנב ר"ל {{#annot:term|1097,1530|CG7S}}מחודד{{#annotend:CG7S}} | ||
| + | |- | ||
| + | |If wishing to examine it, one can look at the examples drawn previously in the chapter of the discussion about the triangle as a principle for all the two-dimensional regular surfaces. | ||
| + | |style="text-align:right;"|וכאשר תרצה לבחון זה עיין בדמיונים המצויירים קודם זה בשער הדבור על שהמשולש פנה לכל בעלי הזויות והקוים הישרים מהשטחים המדותיים | ||
| + | |- | ||
| + | |Again, it is said that the production and increase of every absolute number, as the line, is from the one, such as 1, 2, 3, 4, 5, 6, to that which is added infinitely. | ||
| + | |style="text-align:right;"|ונשוב עתה ונאמר שכל מספר מוגבל כמו הקו הנה תולדתו וצמיחתו מהאחד וזה כמו א' ב' ג' ד' ה' ו' אל מה שיתוסף מזה תמיד | ||
| + | |- | ||
| + | |From the linear numbers the angled surfaces and solids are composed, except that they are not produced and composed accidentally from the construction in thought, but according to a definition and order, as revealed previously when defining the production of the surfaces. | ||
| + | |style="text-align:right;"|ומאלו {{#annot:term|2462,2101|F5O7}}המספרים הקויים{{#annotend:F5O7}} מה שיתרכבו בעלי הזויות מהשטחים והגרמים אלא שלא יתילד ויתרכב איך שיזדמן מההרכבה במחשבה אבל על גבול וסדר כמו שכבר גלינו במה שקדם בהגבילנו צמיחת השטחים | ||
| + | |- | ||
| + | !The production of the solid numbers and their growth | ||
| + | !style="text-align:right;"|ואמנם התילד {{#annot:term|89,1851|wtsx}}המוגשמים{{#annotend:wtsx}} וצמיחתם | ||
| + | |- | ||
| + | | | ||
| + | *The triangular pyramid, i.e. the trianlular solid, is produced by placing one on top, then placing beneath it the triangular numbers that were already mentioned, each beneath its preceeding in rank by order successively. This is done always when wishing to form the triangular pyramid. | ||
| + | |style="text-align:right;"|הנה {{#annot:triangular|1785,2442|nwxw}}המשלש המזדנב{{#annotend:nwxw}} ר"ל הגרם המשלש התילדו בהנחת האחד בגובה עוד תניח תחתיו {{#annot:term|85,1527|aQyD}}המשולשים המספריים{{#annotend:aQyD}} אשר כבר זכרנום כל אחד תחת אשר לפניו במדרגה וסדר כפי המשכם ונפעל זה תמיד מה שנרצה להוליד ה{{#annot:term|1785,2442|lpSn}}מזדנבים{{#annotend:lpSn}} כי יתחדש {{#annot:triangular|1785,1530|XEke}}גוף מחודד משולש{{#annotend:XEke}} | ||
| + | |- | ||
| + | | | ||
| + | *The square pyramids are generated by arranging the aforementioned numerical square surfaces, placing one as their first, then placing beneath it the squares by order successively, and from this a square pyramid is generated. | ||
| + | |style="text-align:right;"|ואמנם {{#annot:square|1785,2442|umoL}}המרובעים המזדנבים{{#annotend:umoL}} ר"ל ה{{#annot:term|1097,1530|6x7Y}}מחודדים{{#annotend:6x7Y}} הנה הם יתחדשו כאשר תסדר אותם {{#annot:term|86,1263|hsY3}}השטחים המרובעים המספריים{{#annotend:hsY3}} אשר זכרנום לפנים והנחת האחד ראשון להם עוד תשים המרובעים תחתיו ימשכו קצתם לקצת יתחדש מזה {{#annot:square|1785,2442|weJa}}מרובע מזדנב{{#annotend:weJa}} | ||
| + | |- | ||
| + | |Likewise for the pentagonal pyramid, the hexagonal pyramid, the heptagonal pyramid, and what is similar to these. | ||
| + | |style="text-align:right;"|וכן המחומש והמשושה והמשובע ומה שידמה זה | ||
| + | |- | ||
| + | | | ||
| + | *The triangular pyramids - the ranks of their formation are as the sequence of these numbers: 1, 4, 10, 20, 35, 56. | ||
| + | |style="text-align:right;"|הנה {{#annot:triangular|1785,2442|OD7J}}הגרמיים המזדנבי' המשולשים{{#annotend:OD7J}} ר"ל המחודדים יהיו מדרגות חדושם כפי משך אלו המספרים א' ד' י' כ ל"ה נ"ו | ||
| + | |- | ||
| + | | | ||
| + | :Each of these numbers is produced from the arrangement of the numbers one beneath the other from one onward as you wish. | ||
| + | |style="text-align:right;"|הנה כל אחד מאלו המספרי' אמנם יתילד מסדר המספרים קצתם תחת קצת מאחד האחד עוד האחד עד אשר תרצה | ||
| + | |- | ||
| + | | | ||
| + | :*One is the potential triangular pyramid | ||
| + | |style="text-align:right;"|ואמנם האחד הנה הוא {{#annot:triangular|1785,1530|gfTU}}גרם המחודד המשולש{{#annotend:gfTU}} בכח | ||
| + | |- | ||
| + | | | ||
| + | :*Four is the second, which is a construction of two triangles - one and three | ||
| + | ::<math>\scriptstyle{\color{blue}{4=1+3}}</math> | ||
| + | |style="text-align:right;"|ואמנם הארבעה הנה הוא השיני והוא הרכבת שני משולשים האחד והשלשה | ||
| + | |- | ||
| + | | | ||
| + | :*ten is a construction of three triangles - one, three and six | ||
| + | ::<math>\scriptstyle{\color{blue}{10=1+3+6}}</math> | ||
| + | |style="text-align:right;"|ואמנם העשרה הוא מהרכבת שלשה משולשים אחד ושלשה וששה | ||
| + | |- | ||
| + | | | ||
| + | :And so the rest in this order are found by this description. | ||
| + | |style="text-align:right;"|וכן הנשארים כפי זה הסדר נמצא בזה התאר | ||
| + | |- | ||
| + | | | ||
| + | *The ranks of the formation of the square pyramids in their succession are as the sequence of these numbers: 1, 5, 14, 30, 55. | ||
| + | |style="text-align:right;"|ואמנם מדרגות התחדש {{#annot:square|1785,1530|4Db7}}הגרמים המרובעים המחודדים{{#annotend:4Db7}} כפי המשכם הנה על משך אלו המספרים א' ה' י"ד ל' נ"ה | ||
| + | |- | ||
| + | | | ||
| + | :Each of these numbers is produced from the construction of the simple squares introduced above, one upon the other, as said about the the triangular pyramid. | ||
| + | |style="text-align:right;"|כי כל אחד מאלו המספרים אמנם התילד מהרכבת המרובעים הפשוטים אשר אמרנו עליהם לפנים קצתם על קצת כמו שאמרנו במשולש המחודד | ||
| + | |- | ||
| + | |As described in this example, so are the pentagonal pyramid, the hexagonal pyramid and others. | ||
| + | |style="text-align:right;"|וכפי זה המשל אשר תארנו יהיה {{#annot:pentagonal|1785,1530|eLrX}}המחומש המחודד{{#annotend:eLrX}} והמשושה וזולתם | ||
| + | |- | ||
| + | |It is clarified from all that was noted, that the units that are in each of the sides of the pyramids are equal to the number of the surfaces arranged in them, whatever they may be, from the one to the surface of the base. | ||
| + | |style="text-align:right;"|ומבואר מכל מה שזכרנו שכל צלע מצלעות מנין המחודדים יחד שוה מה שבם מהאחדים למנין השטחים המסודרים בם היו מה שהיו מהאחד עד שיגיע אל שטח התושבת | ||
| + | |- | ||
| + | |It should not be left out from all that was mentioned, that the simple triangle is the principle and the root of all the surfaces. | ||
| + | |style="text-align:right;"|ולא יעלם ממה שזכרנו שהמשולש הפשוט הוא הפנה והשרש לכל השטחים | ||
| + | |- | ||
| + | |The triangular pyramid is the principle of all pyramids. | ||
| + | |style="text-align:right;"|ו{{#annot:triangular|1097,1530|xeb3}}המשולש המוגשם המחודד{{#annotend:xeb3}} הוא הפנה לכל המחודדים | ||
| + | |- | ||
| + | |After mentioning the pyramids among the solids, now the one that is called truncated is discussed, which is every angular solid, whose arrangement and construction are according to the arrangement of the construction of the pyramids, except that its height does not end in one, which is the apex of the pyramid, and the angles of its top base are equal in number to the angles of its bottom base, this species is called the truncated. | ||
| + | |style="text-align:right;"|ואחר שכבר הבאנו בזכירת המזונב מהמוגשמים נדבר על אשר נקראהו {{#annot:term|2481,1674|EfLj}}מחוסר{{#annotend:EfLj}} והוא כל גשם בעל זויות היה בסדורו והרכבתו על סדר הרכבת המחודדים אלא שלא יכלה בגבהו אל האחד אשר הוא עליון שבמחודד והיו זויות שטח עליונו שוות במספר לזויות שטח תושבתו הנה זה המין יקרא המחוסר | ||
| + | |- | ||
| + | | | ||
| + | *If the pyramid is deficient by one alone, which is apex of the pyramid, it is called uni-truncated. | ||
| + | |style="text-align:right;"|וזה אם היה חסר מהמחודד באחד לבד אשר הוא עליון המחודד יקרא מחוסר אחד | ||
| + | |- | ||
| + | | | ||
| + | *If it is deficient by one surface [= one + the first actual polygon], it is called bi-truncated. | ||
| + | |style="text-align:right;"|ואם היה חסר מהאחד שטח אחד יקרא מחוסר שנים | ||
| + | |- | ||
| + | | | ||
| + | *If it is deficient by a third surface [= one + the first actual polygon + the second actual polygon], it is called tri-truncated. | ||
| + | |style="text-align:right;"|ואם היה חסר שטח שלישי יקרא מחוסר שלשה | ||
| + | |- | ||
| + | | | ||
| + | *If it is deficient by four surfaces [= one + the first actual polygon + the second actual polygon + the third actual polygon], it is called quadri-truncated. | ||
| + | |style="text-align:right;"|וכן המחוסר ארבעה אם היה חסר ארבעה שטחים | ||
| + | |- | ||
| + | |So it is always found. | ||
| + | |style="text-align:right;"|וכן תמצאהו תמיד | ||
| + | |- | ||
| + | |Since the production of this truncated solid is already clarified, as there is no difference between it and what was already explained before about the construction of the pyramid, except that a surface or surfaces are subtracted from the apex of the pyramid, which is the one, this is whole of the discussion. | ||
| + | |style="text-align:right;"|ואחר שכבר התבאר איך צמיחת זה {{#annot:term|2481,1674|fXNB}}הגשם המחוסר{{#annotend:fXNB}} אחר שאין הפרש בינו ובין מה שבארנו לפנים מהרכבת {{#annot:term|1097,1530|pjvt}}המוגשם המחודד{{#annotend:pjvt}} אלא שאנו נחסרהו מעליון המחודד אשר הוא האחד שטח או שטחים הנה זה כלל המאמר על זה | ||
| + | |- | ||
| + | |This is the time to begin the discussion on the rest of the aforementioned solids, which are the cubes, the bricks, the wedges, the circulars, the sphericals, the scalene, and the heteromecic, while endeavoring to explain it, with the help of God. | ||
| + | |style="text-align:right;"|ואמנם המאמר על שאר הגרמים אשר זכרנום לפנים והם המעוקבים וה{{#annot:term|94,2114|IcRl}}לבנים{{#annotend:IcRl}} וה{{#annot:term|2133,2433|Lxft}}אריחיים{{#annotend:Lxft}} וה{{#annot:term|95,2441|SN4f}}קשתיים{{#annotend:SN4f}} וה{{#annot:term|96,2435|gdXU}}כדוריים{{#annot:end||SiHs}} ו{{#annot:term|92,1865|NLH5}}המתחלפי הצלעות{{#annotend:NLH5}} ו{{#annot:term|93,2115|jVUX}}הזולתיים באורך{{#annotend:jVUX}} הנה זה עת להתחיל בו ונשתדל לפרשו בג"ה | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | ====== The Discussion on the Cube Number ====== | ||
| + | |||
| + | |style="text-align:right;"|<big>הדבור במספר המעוקב</big> | ||
| + | |- | ||
| + | |The square equilateral plane numbers discussed above, such as 1, 4, 9, 16, 25, 36, when each one of these surfaces is composed with a surface similar to it in quantity, so that the number of the surfaces composed with each other is as the units of the length and the breadth of the base surface, it is called a cube number. | ||
| + | |style="text-align:right;"|ונאמר עתה שהמספרים המרובעים השוים המשוטחים אשר דברנו עליהם לפנים והם כמו א' ד' ט' י"ו כ"ה ל"ו כאשר הורכב כל אחד מאלו השטחים לדומה לו מהשטחים בכמות עד שיהיה מספר השטחים המורכב קצתם על קצת כמו אחדי אורך ורחב שטח התושבת יקרא מעוקב | ||
| + | |- | ||
| + | |The rule of the discussion: the cube is that whose length, breadth and depth are equal to each other. | ||
| + | |style="text-align:right;"|וכלל המאמר כי המעוקב הוא אשר ארכו ורחבו ועמקו שוה קצתו לקצתו | ||
| + | |- | ||
| + | | | ||
| + | *The four, which is an equilateral number, each side of it is two <math>\scriptstyle{\color{blue}{4=2\times2}}</math>, when four units are composed with them <math>\scriptstyle{\color{blue}{4+4=8}}</math>, the sum is eight, eight is two by two by two <math>\scriptstyle{\color{blue}{8=2\times2\times2}}</math>, and this is equal in the three dimension, i.e. the length, breadth and depth. | ||
| + | |style="text-align:right;"|כי הארבעה אשר הם מספר שוה כל צלע ממנו שנים כאשר הורכב עליהם ארבעה אחדים היה כלל שמנה ושמנה שנים בשנים עוד בשנים וזה שוה המרחקים בשלשה ר"ל האורך והרחב והעמק | ||
| + | |- | ||
| + | | | ||
| + | *The nine, each side of which is three <math>\scriptstyle{\color{blue}{9=3\times3}}</math>, when two surfaces equal to them are composed with them, the sum is 27 <math>\scriptstyle{\color{blue}{9+9+9=27}}</math>, which is the product of three by three by three <math>\scriptstyle{\color{blue}{27=3\times3\times3}}</math> | ||
| + | |style="text-align:right;"|וכן גם כן התשעה אשר כל צלע מצלעיותיו שלשה כאשר הרכבו עליהם שני שטחים אחרים שוים להם היה הכלל כ"ז הוא הגעת מוכה ג' בג' עוד בג‫' | ||
| + | |- | ||
| + | |So for the rest of the cube numbers according to this description. | ||
| + | |style="text-align:right;"|וכן שאר המספרים המעוקבים כפי זה התאר | ||
| + | |- | ||
| + | |Their general rule: each number is multiplied by the measure of its units, then the product is multiplied again by the measure of the units of the first number, so the total sum is a cube number. | ||
| + | |style="text-align:right;"|והכלל הכולל בהם שכל מספר נכפל בשעור אחדיו עוד נכפל הכל בשעור אחדי המספר הראשון שנית הנה הכלל יהיה מספר מעוקב | ||
| + | |- | ||
| + | |From these it is known that any plane square is a surface that has four angles and four sides. | ||
| + | |style="text-align:right;"|הנה כבר קרה מזה שנדע שכל מרובע פשוט הוא שטח ולו ארבע זויות וארבע צלעות | ||
| + | |- | ||
| + | |Any cube has six equal surfaces, eight equal angles, and twelve equal sides. | ||
| + | |style="text-align:right;"|וכל מעוקב לו ששה שטחים שוים ושמנה זויות שוות ושתים עשרה צלעות שוות | ||
| + | |- | ||
| + | |It is said that the sides, the angles, the surfaces, and the lines of the cube are in a harmonic proportion. | ||
| + | |style="text-align:right;"|ואמנם מה שנאמר בו כי צלעות המעוקב וזויותיו ושטחיו וצלעותיו ר"ל קוויו ב{{#annot:term|1771,2095|VOB6}}יחס חבוריי{{#annotend:VOB6}} | ||
| + | |- | ||
| + | | | ||
| + | *The ratio of the surfaces, which are 6, to the angles, which are 8, is a sesquitertian ratio <math>\scriptstyle{\color{blue}{8:6=1+\frac{1}{3}}}</math> | ||
| + | |style="text-align:right;"|וזה שיחס השטחים והם ששה אל הזויות והם שמנה ביחס המוסיף שליש | ||
| + | |- | ||
| + | | | ||
| + | *The ratio of the angles, which are 8, to the sides, which are 12, is a sesquialter ratio <math>\scriptstyle{\color{blue}{12:8=1+\frac{1}{2}}}</math> | ||
| + | |style="text-align:right;"|ויחס הזויות והם שמנה אל הצלעות והם י"ב ביחס המוסיף חצי | ||
| + | |- | ||
| + | | | ||
| + | *The ratio of the surfaces, which are 6, to the sides, which are 12, is a double ratio <math>\scriptstyle{\color{blue}{12:6=2}}</math> | ||
| + | |style="text-align:right;"|הנה יחס השטחים והם ששה אל הצלעות והם שתים עשרה ביחס הכפל השניי | ||
| + | |- | ||
| + | |These two ratios, i.e. the superparticular ratio and the multiple ratio, are the species of the simple ratios, as already clarified above. | ||
| + | |style="text-align:right;"|ושני אלו היחסים ר"ל המוסיף חלק והכפל הם מיני היחסים הפשוטים החבורים כמו שכבר התבאר זה לפנים | ||
| + | |- | ||
| + | |{{#annot:definition|91,1828|YZXv}}Hence, the solid number whose dimensions are equal, i.e. the length, breadth and depth, is necessarily the cube number. | ||
| + | |style="text-align:right;"|הנה אם כן המספר הגרמי השוה המרחקים ר"ל האורך והרחב והעומק הוא המעוקב בהכרח{{#annotend:YZXv}} | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ====== The Discussion on the Scalene Numbers ====== | ||
| + | |||
| + | |style="text-align:right;"|<big> הדבור במספרים הגרמיים המתחלפי המרחקים השלשה</big> | ||
| + | |- | ||
| + | |Now we should discusses the solid that is opposed to the cube, that is, the unequal in all dimensions, i.e. whose length differs from its breadth, and both together differ from its height. | ||
| + | |style="text-align:right;"|וממה שראוי שנדבר עתה הוא בגרם אשר יקביל המעוקב והוא המתחלף המרחקים יחד ר"ל אשר ארכו מתחלף לרחבו והם יחד מתחלפים לגבהו | ||
| + | |- | ||
| + | |For example: breadth = 2, length = 2, height = 4; or length = 3, breadth = 4, depth = 5. | ||
| + | |style="text-align:right;"|משל זה שיהיה הרחב ב' והאורך ג' והגובה ד' או יהיה האורך ג' והרחב ד' והעומק ה‫' | ||
| + | |- | ||
| + | |Or other modes of diversity of the excess of some sides over others. | ||
| + | |style="text-align:right;"|או זולת זה מאופני החלוף בתוספת קצת הצלעות על קצת | ||
| + | |- | ||
| + | |The rule of this discussion is that none of its dimensions is equal to another dimension. | ||
| + | |style="text-align:right;"|וכלל המאמר שלא יהיה דבר ממרחקיו שוה למרחק אחר ממנו | ||
| + | |- | ||
| + | |This number is called by many names: | ||
| + | |style="text-align:right;"|וזה המספר יקרא בשמות רבים | ||
| + | |- | ||
| + | | | ||
| + | *Some people call it scale-like, as it increases from its breadth to its length and from its length to its height. | ||
| + | |style="text-align:right;"|מהם שאנשים יקראוהו ה{{#annot:term|92,2445|m7iX}}סולמיי{{#annotend:m7iX}} מפני שיעלה בהוספה מרחבו לארכו ומארכו לגבהו | ||
| + | |- | ||
| + | | | ||
| + | *Other call it wedge, because of the similarity to the wedge that is a solid of different surfaces in its three dimensions. | ||
| + | |style="text-align:right;"|ויקראהו אחרים הארחיי להתדמות לאריח שהוא {{#annot:term|587,1445|3czY}}גוף{{#annotend:3czY}} מתחלף השטחים בשלשת מרחקיו | ||
| + | |- | ||
| + | |This is the image of their shape and their order according to their natural succession: | ||
| + | |style="text-align:right;"|וזה לך דמיון תמונתם וסדורם כפי המשכם בסדר הטבע | ||
| + | |- | ||
| + | | | ||
| + | *Set a table breadthwise, i.e. the ranks of the breadth of the table are divided according to the ranks of the numbers according to their natural succession. | ||
| + | |style="text-align:right;"|תניח לוח הולך ברחב ר"ל רחב הלוח נרשם מחולק במדרגותיו מדרגות המספרים כפי סדרם בטבע | ||
| + | |- | ||
| + | | | ||
| + | *Set beneath it a second table that is one less rank on the side of the beginning of the ranks, placing in each of its ranks the product of the multiplication of what is above it in the first table by its predecessor. | ||
| + | |style="text-align:right;"|ונניח תחתיו לוח שני יותר חסר ממנו במדרגה מצד התחלת המדרגות עוד נעמיד בכל מדרגה ממנו מה שיתקבץ מהכאת אשר למעלה ממנו מהלוח הראשון עם אשר לפניו | ||
| + | |- | ||
| + | | | ||
| + | *Set beneath them a third table, like the second table and the number of its ranks, placing beneath each rank of the table the product of the multiplication of the rank above it by the third rank of the first table above it, until the third line is completed by this, and all of its ranks are scalene numbers according to their natural succession, without missing any of them by emptying two of their ranks [?]. | ||
| + | |style="text-align:right;"|ותניח גם כן תחתיהם לוח שלישי כמו הלוח השני וכפי מנין מדרגותיו ונעמיד בו תחת כל מדרגה מהלוח מה שיתקבץ ממדרגה אשר עליה ומהכאתה במדרגה השלישית אשר עליה מהלוח הראשון עד שישלם בזה הטור השלישי ויהיו מדרגותיו כלם הם {{#annot:term|92,2445|wKV4}}מספרי הגרמים הסולמיים{{#annotend:wKV4}} כפי המשכם בטבע מבלתי שיחטא בם באחד מהם בפנוי ב' ממדרגותיהם | ||
| + | |- | ||
| + | |Examine it in this diagram written here: | ||
| + | |style="text-align:right;"|ותבחן זה בזאת הצורה אשר רשמנו בכאן | ||
| + | |} | ||
| + | |||
| + | {|class="wikitable" style="margin-left: auto; margin-right: auto; text-align:center;" | ||
| + | |- | ||
| + | |יא||י||ט||ח||ז||ו||ה||ד||ג||ב||א | ||
| + | |- | ||
| + | |קי||צ||עב||נו||מב||ל||כ||יב||ו||ב|| | ||
| + | |- | ||
| + | | ||תתקץ||תשך||תקד||שלו||רי||קכ||ס||כד||ו|| | ||
| + | |} | ||
| + | {|class="wikitable" style="color:blue; margin-left: auto; margin-right: auto; text-align:center;" | ||
| + | |- | ||
| + | |11||10||9||8||7||6||5||4||3||2||1 | ||
| + | |- | ||
| + | |110||90||72||56||42||30||20||12||6||2|| | ||
| + | |- | ||
| + | | ||990||720||504||336||210||120||60||24||6|| | ||
| + | |} | ||
| + | |||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ====== The Discussion on the Parallelepipedon Numbers ====== | ||
| + | |||
| + | |style="text-align:right;"|<big>הדבור במספרים הגרמים הזולתיים</big> | ||
| + | |- | ||
| + | |Since these two species, i.e. the cube and the scalene numbers correspond to the parallelism of the two extremes, as the cube is equal in its three sides, and the other is unequal in its three sides, the mean between these two are the solid numbers that are called parallelepipedons, i.e. their surfaces are heteromecic. | ||
| + | |style="text-align:right;"|ואחר שהיו שני אלו המינים ר"ל המעוקב והמתחלף הצלעות יוקבלו בהקבלת שני הקצוות אחר שהמעוקב שוה הצלעות השלשה והאחר מתחלף הצלעות השלשה הנה האמצעי בין שני אלו הם המספרים הגרמיים אשר יקראו {{#annot:term|93,2115|NGVt}}הזולתיים האורך{{#annotend:NGVt}} ר"ל אשר פשוטיהם זולתיי האורך | ||
| + | |- | ||
| + | |As will soon be stated, the heteromecic number is the number whose measure is defined in a plane as a quadrangular right-angled shape, whose breadth is shorter than its length by one, such as: 2, 6, 12, 20, 30, 42 and their similar. | ||
| + | |style="text-align:right;"|הנה {{#annot:term|1772,2115|Rs4e}}המספר הזולתי האורך{{#annotend:Rs4e}} כבר יאמר שהוא המספר אשר ירשם שעורו בפשוט רושם מרובע נצב הזויות ורחבו פחות מארכו אחד כמו ב' ו' י"ב כ' ל' מ"ב והדומה להם | ||
| + | |- | ||
| + | |When each of these heteromecic surfaces is given a height that is same as one of its sides - the parallelepipedon numbers are produced. | ||
| + | |style="text-align:right;"|הנה כאשר שמנו לכל אחד מאלו הפשוטים הזולתיי האורך גובה באחד מצלעותיו יתילדו מזה {{#annot:term|93,2115|Flvx}}המספרים הגרמיים הזולתיים{{#annotend:Flvx}} | ||
| + | |- | ||
| + | |This is an image of their formation: | ||
| + | |style="text-align:right;"|וזה לך דמיון הנחתם | ||
| + | |- | ||
| + | | | ||
| + | *Two lines - the first and the second - are of the three previous line in the chapter on the extraction of the scalene numbers. | ||
| + | |style="text-align:right;"|שני טורים מהם הראשון והשני הם משלשת הטורים הקודמים בשער הוצאת {{#annot:term|92,2445|4Hc8}}המספרים הגרמיים הסולמיים{{#annotend:4Hc8}} | ||
| + | |- | ||
| + | | | ||
| + | *Multiplying each of the ranks of the second line by each of those that are above it in the first line, i.e. those that it results from their multiplication by each other. | ||
| + | |style="text-align:right;"|ונכה כל מדרגה ממדרגות הטור השני מהם בכל אחת מאשר עליה בטור הראשון ר"ל אשר עמדה מהכאת אחת מהן באחרת | ||
| + | |- | ||
| + | | | ||
| + | *Placing each of the two products in two different ranks, the smaller of them precedes the greater, and both are beneath the product rank of the second line. | ||
| + | |style="text-align:right;"|ונעמיד שני הכללים בשתי מדרגות נבדלות הקטנה מהן קודמת לגדולה ושתיהן תחת המדרגה הנעשית בהכאה מהטור השני | ||
| + | |- | ||
| + | | | ||
| + | *When this table is completed by this technique, the ranks set at the third line are twice as many as the ranks of the second line. | ||
| + | |style="text-align:right;"|וכאשר תשלים הלוח בזאת המלאכה הנה כבר שמת מדרגות הטור השלישי כפל מדרגות הטור השני | ||
| + | |- | ||
| + | |Thus, all parallelepipedon numbers are reproduced according to their natural succession, none of them is missing. | ||
| + | |style="text-align:right;"|וכבר העמדנו בו כל {{#annot:term|93,2115|YQPN}}המספרים הגרמים הזולתיים{{#annotend:YQPN}} כפי המשכם בסדר הטבע לא יבצר מהם אחד | ||
| + | |} | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: auto; text-align:center;" | ||
| + | |- | ||
| + | |colspan="2"|י||colspan="2"|ט||colspan="2"|ח||colspan="2"|ז||colspan="2"|ו||colspan="2"|ה||colspan="2"|ד||colspan="2"|ג||colspan="2"|ב||א | ||
| + | |- | ||
| + | |colspan="2"|צ||colspan="2"|עב||colspan="2"|נו||colspan="2"|מב||colspan="2"|ל||colspan="2"|כ||colspan="2"|יב||colspan="2"|ו||colspan="2"|ב|| | ||
| + | |- | ||
| + | |תתק||תתי||תרנב||תקעו||תמח||שצב||רצד||רנב||קף||קנ||ק||פ||מח||לו||יח||יב||ד||ב|| | ||
| + | |} | ||
| + | {|class="wikitable" style="color:blue; margin-left: auto; margin-right: auto; text-align:center;" | ||
| + | |- | ||
| + | |colspan="2"|10||colspan="2"|9||colspan="2"|8||colspan="2"|7||colspan="2"|6||colspan="2"|5||colspan="2"|4||colspan="2"|3||colspan="2"|2||1 | ||
| + | |- | ||
| + | |colspan="2"|90||colspan="2"|72||colspan="2"|56||colspan="2"|42||colspan="2"|30||colspan="2"|20||colspan="2"|12||colspan="2"|6||colspan="2"|2|| | ||
| + | |- | ||
| + | |900||810||652||576||448||392||294||252||180||150||100||80||48||36||18||12||4||2|| | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | |If some of the sides exceed some of them by more than one, i.e. by two, or three, or four, such as <math>\scriptstyle{\color{blue}{2\times4}}</math>, <math>\scriptstyle{\color{blue}{4\times6}}</math>, or <math>\scriptstyle{\color{blue}{5\times7}}</math>, or more than that, it is not called heteromecic number but an oblong number. | ||
| + | |style="text-align:right;"|ואמנם אם יוסיפו קצת הצלעות על קצת ביותר מאחד ר"ל שנים או שלשה או ארבעה כמו שנים בארבעה וארבעה בששה או חמשה בשבעה או מה שלמעלה מזה לא יקרא מה שהוא כן זולתיי האורך אבל יקרא המוסיף האורך | ||
| + | |- | ||
| + | |Know that two of the quadrangular surfaces of the parallelepipedon numbers are parallel squares and the rest of their surfaces, i.e. the four remaining surfaces of any of the solid numbers among them, each of them is a heteromecic number, its four sides are equal, of one measure, and its eight sides are equal, of one measure differing from the measure of the four by one, additional or deficient. | ||
| + | |style="text-align:right;"|ודע שהמספרים הגרמיים הזולתיים יהיו לעולם שני שטחים משטחיהם מרובעים מקבילים ושאר שטחיהם ר"ל הארבעה שטחים הנשארים בכל {{#annot:term|89,2571|VXpF}}מספר גרמיי{{#annotend:VXpF}} מהם הנה כל אחד מהם יהיה זולתיי האורך ויהיו ארבעת צלעותיו שוים על שעור אחד ושמנה מצלעותיו שוים על שעור אחד מתחלף לשעור הארבעה באחד אם מוסיף ואם חסר | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ====== The Discussion on the Natural Root of the Numerical “Sameness” and Numerical “Otherness” ====== | ||
| + | |||
| + | |style="text-align:right;"|<big>הדבור בשורש הטבעיי ל{{#annot:term|86,2514|Bb1J}}הוא הוא{{#annotend:Bb1J}} וה{{#annot:term|1772,2199|Gk5s}}זולתיות{{#annotend:Gk5s}} המספריים</big> | ||
| + | |- | ||
| + | |The "same" is the number, whose breadth is has the value of its length | ||
| + | |style="text-align:right;"|אמנם ההוא הוא הנה הוא המספר אשר רחבו הוא ארכו {{#annot:term|217,1675|6yiz}}בהגעה{{#annotend:6yiz}} | ||
| + | |- | ||
| + | |The root of the "sameness", according to the ancients of the pythagoreans, is 1, since when it is multiplied by 1, the length is the breadth, and the product is each of them not parting from them at all. | ||
| + | |style="text-align:right;"|ואמנם היה שרש ההוא הוא אצל הקדמונים מכת פתאגורוש האחד לפי שהוא כאשר יוכה באחד היה האורך הוא הרחב והמתקבץ הוא כל אחד מהם לא יצא מעצמו כלל | ||
| + | |- | ||
| + | |The square numbers are called "same", as they partake in this conception, since their length is their breadth. | ||
| + | |style="text-align:right;"|עוד נקראו המספרים המרובעים {{#annot:term|86,2514|t76o}}הוא הויים{{#annotend:t76o}} ללקחם הדמוי מזה לפי שארכם הוא רחבם | ||
| + | |- | ||
| + | |The more appropriate among the numbers to be the root of the otherness is the one which does not differ from the above mentioned true fundamental "same", except by one. | ||
| + | |style="text-align:right;"|והיה היותר ראוי מהמספרים להיות שרש ל{{#annot:term|1772,2199|kdOx}}זולתיי{{#annotend:kdOx}} הוא אשר לא ישתנה מההוא הוא האמתי השרשיי הנזכר לפנים אלא באחד | ||
| + | |- | ||
| + | |Therefore, the root of the "other" numbers should be the second number | ||
| + | |style="text-align:right;"|הנה בראוי אם כן יהיה שרש המספרים הזולתיים הוא המספר השניי | ||
| + | |- | ||
| + | |According to this the "same" and the "other" are drawn. | ||
| + | |style="text-align:right;"|הנה כפי זה היו מקישים {{#annot:term|86,2514|Vzbi}}ההוא הוא{{#annotend:Vzbi}} ו{{#annot:term|1772,2199|qOjh}}הזולת{{#annotend:qOjh}} | ||
| + | |- | ||
| + | |It is already clear from what we preceded and explained: | ||
| + | *that the one is particular to the odd numbers, since one is always [the difference] between its two parts. | ||
| + | |style="text-align:right;"|וכבר היה שתבאר ממה שהקדמנו ובארנו שכל נפרד הנה האחד מיוחד לו אחר שבין שני חלקיו האחד לעולם | ||
| + | |- | ||
| + | | | ||
| + | :One of its two parts necessarily exceeds by one. | ||
| + | |style="text-align:right;"|בהכרח הם יעדיף אחד משני חלקיו לאחד | ||
| + | |- | ||
| + | | | ||
| + | *And that the duality is particular to every even numbers, since it is divided into two similar parts. | ||
| + | |style="text-align:right;"|ושכל זוג הנה השניות מיוחד לו אחר שיחלק בשני חלקים דומים נמשלים | ||
| + | |- | ||
| + | |It should be said that the odd number, as it is the root of the "same", is particular for the nature of the "same". | ||
| + | |style="text-align:right;"|ובראוי נאמר כי הנפרד מיוחד אחר שזאת שהוא שורש ההוא הוא מיוחד לו לטבע ההוא הוא | ||
| + | |- | ||
| + | |And the even number is particular for the "other". | ||
| + | |style="text-align:right;"|והזוג מיוחד לטבע הזולת | ||
| + | |- | ||
| + | |Since, from the successive addition of the odd numbers, beginning with the one, the "same" numbers are generated, i.e. the squares, that are equal by the measure of the length and the breadth, as explained a few times. | ||
| + | |style="text-align:right;"|כי בתוספת הנפרדים קצתם על קצת ובהקדים האחד בהנחה ראשונה להם יצמחו מספרי ההוא הוא ר"ל המרובעים השוים במרחק האורך והרחב כמו שבארנו זה פעמים | ||
| + | |- | ||
| + | |And from the successive addition of the natural even numbers, beginning with the two, the "other" numbers are produced. | ||
| + | |style="text-align:right;"|ובתוספת הזוגות הטבעיים קצתם על קצת ובהקדים השנים ראשונה להם בהנחה יתילדו הזולתיים | ||
| + | |- | ||
| + | | | ||
| + | *The numbers that are equal by length and breadth are called the "same" numbers, as they partake the conception of the "sameness" of the one. | ||
| + | |style="text-align:right;"|ואמנם קראנו אלו המספרים השוים באורך וברחב מספרי הוא הוא ללקחם דמוי הוא הוא האחד | ||
| + | |- | ||
| + | | | ||
| + | *The numbers that differ in length and breadth by one are called heteromecic, as they partake the conception of the "duality" that departs from the nature of the true "sameness" by one. | ||
| + | |style="text-align:right;"|וקראנו המתחלפים באורך וברחב באחד {{#annot:term|1772,2199|xyKW}}זולתיים{{#annotend:xyKW}} ללקחם דמוי השניות היוצא מטבע ההוא הוא האמיתי באחד | ||
| + | |- | ||
| + | |When comparing the terms of the "same" numbers and the terms of the "other" numbers in two lines, each term compared to its corresponding, one finds that the excess of the "other" term over the corresponding "same" rank is the number, from which the name the term is derived. | ||
| + | |style="text-align:right;"|וכאשר תקביל בשני טורים בין מדרגות המספרים ההוא הויים ומדרגות המספרים הזולתיים כל מדרגה במקביליה תמצא מותר המדרגה הזולתיית על הנכחיית לה {{#annot:term|86,2514|Zt3n}}ההוא הויית{{#annotend:Zt3n}} הוא המניין אשר ממנו נגזר שם המדרגה | ||
| + | |- | ||
| + | | | ||
| + | :{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | |49||36||25||16||9||4||1 | ||
| + | |- | ||
| + | |42||30||20||12||6||2|| | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | |מט||לו||כה||יו||ט||ד||א | ||
| + | |- | ||
| + | |מב||ל||כ||יב||ו||ב|| | ||
| + | |} | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ====== The Discussion on the Brick Numbers ====== | ||
| + | |||
| + | |style="text-align:right;"|<big>הדבור על {{#annot:term|94,2114|TRZT}}המספרים הלבניים{{#annotend:TRZT}} והמגודרים</big> | ||
| + | |- | ||
| + | |The numbers that are similar to bricks are of a species of the parallelepipedon numbers, in which the measure of their four equal sides is one less than the measure of their eight equal sides. | ||
| + | |style="text-align:right;"|ואמנם {{#annot:term|94,2114|en4P}}המספרים הדומים ללבנים{{#annotend:en4P}} הוא המין מהמספרים הגרמיים הזולתיים אשר יהיה שעור צלעותיו הארבעה השוים פחות אחד משעור צלעותיו השמנה השוים | ||
| + | |- | ||
| + | |Those are the terms whose explanation is written in the column of the parallelepipedon numbers. | ||
| + | |style="text-align:right;"|והם המדרגות הנרשם עליהם באורם בטור המספרים הגרמיים והזולתיים | ||
| + | |- | ||
| + | |These are those whose length and breadth are equal and the height is one less than each of them. | ||
| + | |style="text-align:right;"|והם אשר ארכם ורחבם שוה ויחסר הגובה מכל אחד מהם אחד | ||
| + | |- | ||
| + | |If its height exceeds the length and breadth that are equal by the excess of the "other", i.e. by one, or by the excess of the oblong number, i.e. by more than one, such numbers, one of whose three dimensions exceeds the others, are called beam solids. | ||
| + | |style="text-align:right;"|ואמנם אם יוסיף בגובה על האורך והרחב השוים תוספת הזולתיי ר"ל אחד או תוספת המוסיף באורך ר"ל יותר מאחד היו מה שהיה המספר הנוסף באחד מהמרחקים השלשה הנה כל אלו יקראו {{#annot:term|2434,2433|fZcR}}מוגשמים אריחיים{{#annotend:fZcR}} | ||
| + | |- | ||
| + | |Such as: | ||
| + | :*<math>\scriptstyle{\color{blue}{3\times3\times4}}</math> | ||
| + | |style="text-align:right;"|כמו ג' בג' בד‫' | ||
| + | |- | ||
| + | | | ||
| + | :*<math>\scriptstyle{\color{blue}{5\times5\times6}}</math> | ||
| + | |style="text-align:right;"|או ה' בה' בו‫' | ||
| + | |- | ||
| + | |Or as much as one wishes, as long as the height is longer than both the length and the breadth. | ||
| + | |style="text-align:right;"|או כמה שתרצה אחר שיהיה הגובה יותר ארוך מכל אחד מהאורך והרחב | ||
| + | |- | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ====== The Discussion on the Circular and Spherical Numbers ====== | ||
| + | |||
| + | |style="text-align:right;"|<big>הדבור במספרים ה{{#annot:term|95,2444|UYp2}}סבוביים{{#annotend:UYp2}} והכדוריים</big> | ||
| + | |- | ||
| + | |After describing the quality of the solids whose surfaces are of straight lines: | ||
| + | |style="text-align:right;"|ואחר שכבר הבאנו תאר הגרמים בעלי השטחים הישרי הקוים | ||
| + | |- | ||
| + | |We say, as we said that the cube number is of equal dimensions, i.e. the length, the breadth, and the depth, it is divided into two parts: | ||
| + | |style="text-align:right;"|הנה נאמר כמו שאמרנו שהמספר המעוקב הוא השוה המרחקים ר"ל האורך והרחב והעומק וזה יחלק לשני חלקים | ||
| + | |- | ||
| + | | | ||
| + | *The number that is the root of each of its surfaces, whether mentioned in the total surface and in the total solid repeatedly, and again mentioned in the total solid when multiplied by it once, or twice, or more, | ||
| + | |style="text-align:right;"|אם שיהיה המספר אשר הוא שרש כל אחד משטחיו נזכר בכלל השטח עוד נזכר בכלל הגרם חוזר על עצמו עוד נזכר גם כן בכלל הגרם כאשר הוכה בו פעם או שני פעמים או יותר מזה | ||
| + | |- | ||
| + | | | ||
| + | *or whether not so. | ||
| + | |style="text-align:right;"|ואם שלא יהיה כן | ||
| + | |- | ||
| + | |Those, whose roots are expressed in their total among these cube numbers are called spherical numbers. | ||
| + | |style="text-align:right;"|הנה אשר שרשיהם נזכרים בכללם במבטא הגעתם מאלו המספרים המעוקבים הם אשר יקראו {{#annot:term|96,2435|hHXI}}המספרים הכדוריים{{#annotend:hHXI}} | ||
| + | |- | ||
| + | |Since they revolve, turning back as a sphere, to the number from which they begin. | ||
| + | |style="text-align:right;"|אחר שיסבבו מתהפכים כמו הכדור אל המספר אשר ממנו התחלתם | ||
| + | |- | ||
| + | |Such as the cube number, whose side is 5 or 6, for as much as one multiplies one of them by the same, and the product by its root, it is as described. | ||
| + | |style="text-align:right;"|כמו המספר המעוקב אשר הצלע מצלעותיו חמשה או ששה כי כל מה שתכה אחד משני אלו בכמהו עוד מה שיתקבץ בשרשו יהיה נמצא כמו שתארנו | ||
| + | |- | ||
| + | | | ||
| + | :*<math>\scriptstyle{\color{blue}{5\times5=25}}</math> the five returns, expressed in the total. | ||
| + | |style="text-align:right;"|וזה שאנו אם נכה חמשה בחמשה היה המתקבץ כ"ה וישוב החמשה מבוטא בו בכלל וישוב אליו | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{25\times5=125}}</math> the five is again expressed in the total. | ||
| + | |style="text-align:right;"|עוד נכה החמשה גם כן בכ"ה ותמצא החמשה גם כן מבוטא בו בכללם והוא קכ"ה | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{125\times5}}</math> | ||
| + | |style="text-align:right;"|וכן אם תכה הקכ"ה בחמשה | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(125\times5\right)\times5}}</math> | ||
| + | |style="text-align:right;"|ומה שהתקבץ גם כן בחמשה | ||
| + | |- | ||
| + | | | ||
| + | ::It will be found always with this quality. | ||
| + | |style="text-align:right;"|תמצאהו בזה התאר לעולם | ||
| + | |- | ||
| + | | | ||
| + | :*So also the six in this matter, its rule is as the rule of the five. | ||
| + | |style="text-align:right;"|וכן גם כן הששה בזה הענין משפטם משפט החמשה | ||
| + | |- | ||
| + | | | ||
| + | :*It is also found in the one, since one by the same is the same <math>\scriptstyle{\color{blue}{1\times1=1}}</math> | ||
| + | |style="text-align:right;"|וכן תמצא זה באחד כי האחד בכמהו | ||
| + | |- | ||
| + | | | ||
| + | ::then by the same, and again by the same, always not departing from itself, but returning to itself. | ||
| + | |style="text-align:right;"|עוד בכמוהו עוד בכמהו עוד כן תמיד בלתי יוצא מעצמו אבל סובב על עצמו | ||
| + | |- | ||
| + | |Since what was described is already clear, it is said that the circular number is formed by multiplying the number once by itself: | ||
| + | |style="text-align:right;"|ואחר שכבר התבאר מה שתארנו נאמר ש{{#annot:term|95,2444|qSba}}המספר הסבוביי{{#annotend:qSba}} מזה שתכה המספר פעם אחת בעצמו | ||
| + | |- | ||
| + | | | ||
| + | :*<math>\scriptstyle{\color{blue}{1\times1}}</math> | ||
| + | |style="text-align:right;"|כמו א' בא‫' | ||
| + | |- | ||
| + | | | ||
| + | :*<math>\scriptstyle{\color{blue}{5\times5}}</math> | ||
| + | |style="text-align:right;"|וה' בה‫' | ||
| + | |- | ||
| + | | | ||
| + | :*<math>\scriptstyle{\color{blue}{6\times6}}</math> | ||
| + | |style="text-align:right;"|וו' בו‫' | ||
| + | |- | ||
| + | |If it is multiplied more than once, it is a spherical number. | ||
| + | |style="text-align:right;"|ואם הוכה יותר מפעם הנה הוא {{#annot:term|96,2435|5Dmo}}מספר כדורי{{#annotend:5Dmo}} | ||
| + | |- | ||
| + | |Since the circles are surfaces, and the spheres are solids. | ||
| + | |style="text-align:right;"|אחר שהעגולים פשוטים והכדורים גרמיים | ||
| + | |- | ||
| + | |This is the end of the discussion concerning the solid numbers. | ||
| + | |style="text-align:right;"|וזה סוף המאמר על {{#annot:term|89,2571|Oja6}}המספרים הגרמיים{{#annotend:Oja6}} | ||
| + | |- | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== The Discussion of the Indications of the Ancients that the Beginning of Numbers is similar to the Beginning of the World ==== | ||
| + | |||
| + | |style="text-align:right;"|<big>הדבור ברמזי הראשונים על ההתחלות המספריות הדומות להתחלות העולם</big> | ||
| + | |- | ||
| + | |From what was said before about the "other" numbers follows their difference from the heteromecic numbers. | ||
| + | |style="text-align:right;"|וכבר ימשך למה שאמרנו לפנים על המספרים הזולתיים ההפרש בם מהמספרים המוסיפים באורך | ||
| + | |- | ||
| + | |For we already explained that the "others" are those, either of whose dimension exceeds the other by one. | ||
| + | |style="text-align:right;"|שאנו כבר בארנו כי הזולתיים הוא אשר אחד ממרחקיו השנים יותר מהאחר באחד | ||
| + | |- | ||
| + | |While the heteromecic is that whose production accords with this aspect, but the excess of one of its dimension over the other is greater than one. | ||
| + | |style="text-align:right;"|ואמנם המוסיף הוא מה שהיתה צמיחתו כפי זה הצד אלא שתוספת אחד ממרחקיו על האחר יותר מאחד | ||
| + | |- | ||
| + | | | ||
| + | ::Such as: | ||
| + | :*<math>\scriptstyle{\color{blue}{8=2\times4}}</math> | ||
| + | |style="text-align:right;"|כמו ח' המתקבץ מהכאת ב' בד‫' | ||
| + | |- | ||
| + | | | ||
| + | :*<math>\scriptstyle{\color{blue}{18=3\times6}}</math> | ||
| + | |style="text-align:right;"|וכמו י"ח אשר יתקבץ מהכאת ג' בו‫' | ||
| + | |- | ||
| + | |And those similar to this, where the difference between the two numbers, one of which is multiplied by the other, is more than one. | ||
| + | |style="text-align:right;"|והדומה לזה ממה שהחלוף בו בין שני המספרים אשר יוכה אחד מהם באחר ביותר מאחד | ||
| + | |- | ||
| + | |'''He''' [= Nicomachus] '''said''': because of the difference between these numbers, i.e. the "same", i.e. those that have root and the "others", i.e. those whose length exceeds over their breadth by more than one, the separation, the division, and the divergence were created in the number of the species. | ||
| + | |style="text-align:right;"|<big>אמר</big> ולחלוף אלו המספרים ר"ל ההוא הויים ר"ל השרשיים והזולתיים ר"ל אשר יוסיף ארכם על רחבם יותר מאחד חודשה ההפרדה וההחלק והסתעפות מה שבמספר מהמינים | ||
| + | |- | ||
| + | |'''He''' [= Nicomachus] '''said''': it is appropriate that the ancients began the principles of number by starting the discussion about the nature of the world. | ||
| + | |style="text-align:right;"|<big>אמר</big> ובראוי מה שהתחילו הקדמונים הראשיות במספר בהתחילם המאמר על טבע העולם | ||
| + | |- | ||
| + | |'''Plato''' mentioned that the nature of the world is of three ends: | ||
| + | |style="text-align:right;"|אם אפלאטון הנה זכר שטבע העולם משלשה גבולים | ||
| + | |- | ||
| + | | | ||
| + | :1) the same | ||
| + | |style="text-align:right;"|אחד מהם ההוא הוא | ||
| + | |- | ||
| + | | | ||
| + | :2) the other | ||
| + | |style="text-align:right;"|והשני הזולת | ||
| + | |- | ||
| + | | | ||
| + | :3) the substance that is indivisible | ||
| + | |style="text-align:right;"|והשלישי מהעצם אשר אינו מתחלק | ||
| + | |- | ||
| + | |This statement, may God lead you straight, is far from injustice and of very subtle property. | ||
| + | |style="text-align:right;"|וזה המאמר יישירך השם רחוק העול מאד דק הענין | ||
| + | |- | ||
| + | |'''Plato''' refers in it to the principles of the world and this is a thing that is beyond the art of number. | ||
| + | |style="text-align:right;"|ואפלאטון ירמז בו אל ראשיות העולם וזה דבר חוץ ממלאכת המספר | ||
| + | |- | ||
| + | |Had I not agreed not to mix anything into the science of this book, I would have passed over this chapter, and had I not been sure that it would not be conceived as useless lengthiness, and had I not been confident in your current level in philosophy, I would not have mentioned to you that there is a hint under this discussion, and not that it is far from injustice and of very subtle property. | ||
| + | |style="text-align:right;"|ולולא שאני הסכמתי שלא אערב דבר בחכמת זה הספר עברתי זה השער ולולא שאני בטוח שלא ידומה עלי לאריכות לבלתי תועלת וגם בטחוני במדרגתך היום מהעיון לא זכרתי לך שתחת זה המאמר רמז ולא שהוא רחוק העול ודק הענין | ||
| + | |- | ||
| + | |'''The author of this book''' [= Nicomachus] '''said''': '''Philolaus''' says that all the things that exist are necessarily either limited, or limitless, or limited and limitless together. | ||
| + | |style="text-align:right;"|<big>אמר מניח הספר</big> אמנם פילולאוש יאמר שכל הדברים אשר הם נמצאים הנה הם בהכרח אם מוגבלים נכללים ואם בלתי מוגבלים ואם מוגבלים ובלתי מוגבלים יחד | ||
| + | |- | ||
| + | |It is said: and this third saying is about the manner of the number and its image. | ||
| + | |style="text-align:right;"|אמר וזה המאמר השלישי אמנם הוא מאופן המספר ודמיונו | ||
| + | |- | ||
| + | |For the number is composed of the units, and its species are even and odd, which indicate the equality and inequality, the "sameness" and "otherness", the bound and boundlessness, the definite and indefinite | ||
| + | |style="text-align:right;"|כי המספר מורכב מהאחדים ומיניו זוג נפרד והם מורים על השווי והחלוף וההוא הויים והזולתיים וההקפה ובלתי הקפה וההגדרה ובלתי הגדרה | ||
| + | |- | ||
| + | |What was said is clear. | ||
| + | |style="text-align:right;"|ויהיה מה שנאמר מבואר | ||
| + | |- | ||
| + | |He said that the things that exist are produced and persist by the opposite of different and similar, and what was shown about the composition and harmony is appropriate, since the composition is doubtlessly, as explained in the introduction of the first section of this book, from similar diverse things, as the composition is a mixture of things of diverse definitions and similar sepcies. | ||
| + | |style="text-align:right;"|ואמר שהדברים אשר הם נמצאים התילדו ועמדו מהמתנגדים המתחלפים הדומים ובראוי מה שהראו החבור וההסכמה כי החבור אמנם יהיה בלי ספק כמו שבארנו בפתיחת המאמר הראשון מזה הספר מהדברים המתחלפים הדומים הנמצאים כי החבור אמנם הוא ערוב דברים מתחלפי הגדרים דומי הסוגים | ||
| + | |- | ||
| + | |'''Abū Yusūf''' '''said''': he epxressed this statement and compared the limited things to the numbers, which have roots, that are limited, since their root is expressible, that they are formed after it is counted by it self; and he compared the unlimited to the "other" things that are mute, and their root is inexpressible. | ||
| + | |style="text-align:right;"|<big>אמר אבו יוסף</big> אמנם אמר זה המאמר והוא ימשיל המוגבל מהדברים במספרים הנגדרים שהם מוגבלים אחר ש{{#annot:term|1075,2193|aqYC}}ידובר בגדרם{{#annotend:aqYC}} ר"ל שרשם שאחר שימנה בעצמו יחודש ויראו וימשיל הבלתי מוגבלים מהדברים הזולתיים שהם אלמים {{#annot:term|2370,2193|X6wY}}לא ידובר בשרש{{#annotend:X6wY}} דבר מהם | ||
| + | |- | ||
| + | |He meant by limited and unlimited together the heteromecic, for among them are those that have roots as well as those that have no root. | ||
| + | |style="text-align:right;"|ורצה במוגבל ובלתי מוגבל יחד המוסיף האורך כי ממנו שרשי וממנו בלתי שרשיי | ||
| + | |- | ||
| + | |Those that have a root are limited and those that have no root are unlimited. | ||
| + | |style="text-align:right;"|הנה השרשיי מוגבל ובלתי שרשיי בלתי מוגבל | ||
| + | |- | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== Properties of the species of Numbers and their Mutual Harmony ==== | ||
| + | |||
| + | |style="text-align:right;"|<big>הדבור בקצת סגולות מיני המספרים ומה שיראה בהתילדם והקבלת קצתם לקצת</big> | ||
| + | |- | ||
| + | |We have already brought in the introductory comments what we wanted to preface. | ||
| + | |style="text-align:right;"|כבר הבאנו בהקדמות מה שרצינו להקדימו | ||
| + | |- | ||
| + | |Now, it will be shown how many of those described in the description of the "same" and "other" numbers are produced: | ||
| + | |style="text-align:right;"|ונראה איך יתילדו הרבה ממה שתארנו מתואר המספרים ההוא הויים והזולתיים | ||
| + | |- | ||
| + | | | ||
| + | :*Arranging the square numbers that were produced by summing the natural odd numbers successively, i.e. those with roots, in a line. | ||
| + | |style="text-align:right;"|וזה בשנסדר המספרים המרובעים אשר נולדו בהוספת הנפרדים הטבעיים קצתם על קצת ר"ל הנגדרים בטור | ||
| + | |- | ||
| + | | | ||
| + | :*Arranging the heteromecic numbers, i.e. those that were produced by summing the even numbers, successively in a second line. | ||
| + | |style="text-align:right;"|עוד נסדר הזולתיי האורך ר"ל אשר התילדו מתוספת הזוגות המתילדים קצתם על קצת בטור שני | ||
| + | |- | ||
| + | |For this way of action is truly seen visible in the nature of all. | ||
| + | |style="text-align:right;"|כי זה האופן מן הפועל באמת כבר נראהו גלוי בטבע הכל | ||
| + | |- | ||
| + | |The line according to this example is: | ||
| + | |style="text-align:right;"|ויהיה הטור כפי זה המשל | ||
| + | |- | ||
| + | |} | ||
| + | |||
| + | {|class="wikitable" style="margin-left: auto; margin-right: auto; text-align:center;" | ||
| + | |- | ||
| + | | ||קמד||קכא||ק||פא||סד||מט||לו||כה||יו||ט||ד||א | ||
| + | |- | ||
| + | | ||קנו||קלב||קי||צ||עב||נו||מב||ל||כ||יב||ו||ב | ||
| + | |- | ||
| + | |התוספת||יב||יא||י||ט||ח||ז||ו||ה||ד||ג||ב||א | ||
| + | |} | ||
| + | {|class="wikitable" style="color:blue; margin-left: auto; margin-right: auto; text-align:center;" | ||
| + | |- | ||
| + | | ||144||121||100||81||64||49||36||25||16||9||4||1 | ||
| + | |- | ||
| + | | ||156||132||110||90||72||56||42||30||20||12||6||2 | ||
| + | |- | ||
| + | |addition||12||11||10||9||8||7||6||5||4||3||2||1 | ||
| + | |} | ||
| + | |||
| + | {| | ||
| + | |- | ||
| + | |It is clear from the wonders of the nature's action that the first of the "others" is in the double ratio to the first of the "same"; the second to the second are in the sesquialter ratio; the third to the third are in the sesquitertian ratio; the fourth to the fourth are in the sesquiquartian ratio; and so on, one always finds them together according to this description. | ||
| + | |style="text-align:right;"|והוא מבואר מפליאות פועל הטבע שהראשון מהזולתיים ביחס הכפל השניי אצל הראשון מן ההוא הויים וכשני אל השני ביחס המוסיף חצי והשלישי אל השלישי ביחס המוסיף שלישי וכן הרביעי אל הרביעי ב{{#annot:sesquiquartan|125|WiHa}}יחס המוסיף רביע{{#annotend:WiHa}} וכן תמצאם תמיד יחד בכמו זה התאר | ||
| + | |- | ||
| + | |What was said afore is also clarified in these two lines, that if the excess of each term of the "others" over its counterpart among the "same" is taken and set in a third line successively, the terms are found to be the numbers by the natural succession. | ||
| + | |style="text-align:right;"|והתבאר גם כן בשני אלו הטורים מה שכבר אמרנו במה שקדם שאנו אם לקחנו תוספת כל מדרגה מהזולתיים על נכחה מהמדרגות ההוא הויים והנחנוהו בטור שלישי כפי המשכיה מצאנו מדרגות זה הם המספרים על סדר הטבע | ||
| + | |- | ||
| + | |This is found also by: | ||
| + | :*Taking the terms of the "same", beginning with four. | ||
| + | |style="text-align:right;"|וכן תמצאהו כאשר תתחיל במדרגות ההוא הויים ותשים ראש מדרגותיהם ארבעה | ||
| + | |- | ||
| + | | | ||
| + | :*Adding the excess of each term among them over its counterpart among the terms of the "others". | ||
| + | |style="text-align:right;"|עוד תוסיף תוספת כל מדרגה מהם על נכחה ממדרגות הזולתיי | ||
| + | |- | ||
| + | | | ||
| + | :*Arranging them in a third line successively - they are found in the succession of the natural numbers. | ||
| + | |style="text-align:right;"|עוד תסדרם בטור שלישי כפי המשכיהם תמצא זה בסדר המספרים הטבעיים | ||
| + | |- | ||
| + | | | ||
| + | :*The excess of 4 over 2 is 2. <math>\scriptstyle{\color{blue}{4-2=2}}</math> | ||
| + | |style="text-align:right;"|כי תוספת ד' על ב' ב‫' | ||
| + | |- | ||
| + | | | ||
| + | :*The excess of 9 over 6 is 3. <math>\scriptstyle{\color{blue}{9-6=3}}</math> | ||
| + | |style="text-align:right;"|ותוספת ט' על ו' ג‫' | ||
| + | |- | ||
| + | | | ||
| + | :And so on, one finds it always according to this description. | ||
| + | |style="text-align:right;"|וכן תמצאהו תמיד בזה התאר | ||
| + | |- | ||
| + | | | ||
| + | :{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | | ||72||56||42||30||20||12||6||2 | ||
| + | |- | ||
| + | | ||81||64||49||36||25||16||9||4 | ||
| + | |- | ||
| + | |addition||9||8||7||6||5||4||3||2 | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | | ||עב||נו||מב||ל||כ||יב||ו||ב | ||
| + | |- | ||
| + | | ||פא||סד||מט||לו||כה||יו||ט||ד | ||
| + | |- | ||
| + | |התוספת||ט||ח||ז||ו||ה||ד||ג||ב | ||
| + | |} | ||
| + | |- | ||
| + | |If you take their ratio, if they accord with this assumption and you wish to take their ratio, you will find them in the same ratios by which they were seen previously: | ||
| + | |style="text-align:right;"|ואמנם אם תקיש ביניהם והם כפי זאת ההנחה ותרצה ליחסם תמצאם באותם היחסים אשר נראו בראשונה בעצמם | ||
| + | |- | ||
| + | | | ||
| + | :*4 to 2 is in double ratio. <math>\scriptstyle{\color{blue}{4=2\sdot2}}</math> | ||
| + | |style="text-align:right;"|וזה שד' אצל ב' בייחסם הכפל השניי | ||
| + | |- | ||
| + | | | ||
| + | :*9 to 6 is in sesquialter ratio. <math>\scriptstyle{\color{blue}{9=6+\left(\frac{1}{2}\sdot6\right)}}</math> | ||
| + | |style="text-align:right;"|וט' אצל ו' ביחס המוסיף חצי | ||
| + | |- | ||
| + | | | ||
| + | :*16 to 12 is in sesquitertian ratio. <math>\scriptstyle{\color{blue}{16=12+\left(\frac{1}{3}\sdot12\right)}}</math> | ||
| + | |style="text-align:right;"|וי"ו אצל י"ב ביחס המוסיף שליש | ||
| + | |- | ||
| + | | | ||
| + | :Likewise it is found always according to the first property. | ||
| + | |style="text-align:right;"|וכמו זה תמצאהו תמיד על צד הענין הראשון | ||
| + | |- | ||
| + | |It is clear that the excess of the "same" to each other are the natural odds | ||
| + | |style="text-align:right;"|ואמנם תוספת מדרגות ההוא הויים קצתם על קצת הוא מבואר שהם הנפרדים הטבעים | ||
| + | |- | ||
| + | |and the natural odds and natural evens and the multiple ratio and the superparticular ratio [apparently broken sentence] | ||
| + | |style="text-align:right;"|והנפרדים הטבעיים והזוגות הטבעיים ויחס הכפל ויחס המוסיף חלק | ||
| + | |- | ||
| + | | | ||
| + | ::*Setting the first of the "others" as mean between the first two of the squares, i.e. two between one and four. <math>\scriptstyle{\color{blue}{1;\;{\color{red}{2}};\;4}}</math> | ||
| + | |style="text-align:right;"|ואמנם אם תניח הראשון מהזולתיים אמצעי בין שני הראשונים מן המרובעים ר"ל שנים בין אחד וארבעה | ||
| + | |- | ||
| + | | | ||
| + | ::*Setting the second of the "others" as mean between the consecutive of the squares, i.e. six between four and nine. <math>\scriptstyle{\color{blue}{4;\;{\color{red}{6}};\;9}}</math> | ||
| + | |style="text-align:right;"|ותניח השני מן הזולתיים אמצעי בין אשר אחריהם מן המרובעים ר"ל ו' בין ד' וט‫' | ||
| + | |- | ||
| + | | | ||
| + | ::*[Setting] the third "other" between two other squares, following those that were set. | ||
| + | |style="text-align:right;"|וכן השלישי הזולתיי בין שני המרובעים אחרים אחר אשר הנחת | ||
| + | |- | ||
| + | | | ||
| + | ::Proceeding likewise for each term of the "others" successively. | ||
| + | |style="text-align:right;"|וכן תעשה כל מדרגה ממדרגות הזולתיי כפי המשכם | ||
| + | |- | ||
| + | | | ||
| + | ::One finds that it is in the ratio of the terms of the even-times-even numbers | ||
| + | |style="text-align:right;"|תמצא זה ביחס מדרגות זוג הזוג | ||
| + | |- | ||
| + | | | ||
| + | ::The measure of the first to the second is as the measure of the second to the third <math>\scriptstyle{\color{blue}{a_2:a_1=a_3:a_2}}</math> | ||
| + | |style="text-align:right;"|שעור הראשון אצל השני כשעור השני אצל השלישי | ||
| + | |- | ||
| + | | | ||
| + | ::Every three numbers among them, i.e. those whose mean between every two terms of the "same" among them is a term of the "others", according to the explained order, are found in this ratio, i.e. the ratio of the first to the second is as the ratio of the second to the third <math>\scriptstyle{\color{blue}{a_2:a_1=a_3:a_2}}</math>; and vice versa, the third to the second is as the second to the first <math>\scriptstyle{\color{blue}{a_2:a_3=a_1:a_2}}</math>. | ||
| + | |style="text-align:right;"|וכן תמצא כל שלשה מספרים מהם ר"ל מאלו אשר הממצע בין כל שתי מדרגות מההוא הויים מהם מדרגה מהזולתיים כפי סדרם אשר בארנו בזה היחס ר"ל ששעור הראשון מן השני כשעור השני מן השלישי ובהפך גם כן השלישי אצל השני כשני אצל הראשון | ||
| + | |- | ||
| + | | | ||
| + | :It is clear that when these terms are in this order, the excesses of their terms one over the other are unequal. | ||
| + | |style="text-align:right;"|ומבואר שאלו המדרגות כאשר היו כפי זה הסדר לא יהיה תוספת ממדרגותיהם קצתם על קצת שוים | ||
| + | |- | ||
| + | | | ||
| + | :This is their diagram: | ||
| + | |style="text-align:right;"|וזה צורתו | ||
| + | |- | ||
| + | |} | ||
| + | |||
| + | {|class="wikitable" style="margin-left: auto; margin-right: auto; text-align:center;" | ||
| + | |- | ||
| + | |פא||עב||סד||נו||מט||מב||לו||ל||כה||כ||יו||יב||ט||ו||ד||ב||א | ||
| + | |} | ||
| + | {|class="wikitable" style="color:blue; margin-left: auto; margin-right: auto; text-align:center;" | ||
| + | |- | ||
| + | |81||72||64||56||49||42||36||30||25||20||16||12||9||6||4||2||1 | ||
| + | |} | ||
| + | |||
| + | {| | ||
| + | |- | ||
| + | |The property of terms of the even-times-odd numbers is that when they are arranged successively in a special line, they are in the succession of the natural numbers. | ||
| + | |style="text-align:right;"|ואמנם זוג הנפרד הנה סגולת מדרגותיו בסדורם כפי המשכם בטור מיוחד סדור המספרים הטבעיים | ||
| + | |- | ||
| + | |That is, the excesses of the terms of the even-times-odd numbers one over the other are equal: | ||
| + | |style="text-align:right;"|וזה שזוג הנפרד הגעות תוספת מדרגותיו קצתם על קצת שוים | ||
| + | |- | ||
| + | | | ||
| + | :*The excess of 10 over 6 is as the excess of 14 over 10. <math>\scriptstyle{\color{blue}{10-6=14-10}}</math> | ||
| + | |style="text-align:right;"|כי תוספת י' על ו' כמו תוספת י"ד על י‫' | ||
| + | |- | ||
| + | | | ||
| + | :*The excess of 2 over 1 is as the excess of 3 over 2. <math>\scriptstyle{\color{blue}{2-1=3-2}}</math> | ||
| + | |style="text-align:right;"|וכן תוספת ב' על א' כמו תוספת ג' על ב‫' | ||
| + | |- | ||
| + | | | ||
| + | :From these two is understood that double the mean is as the sum of the two extremes <math>\scriptstyle{\color{blue}{2a_2=a_1+a_3}}</math>, and this and its like were already explained in the first section. | ||
| + | |style="text-align:right;"|וישיג שני אלו יחד שיהיה האמצעי נכפל כמו שני הקצוות מקובצים וכבר בארנו זה ודומה לו במאמר הראשון | ||
| + | |- | ||
| + | |The triangular numbers are generated from the combination of the "same" and the "others", each term with its counterpart, successively. | ||
| + | |style="text-align:right;"|ונאמר שהמספרים המשולשים אמנם יתחדשו מהרכבת ההוא הויים והזולתיים כל מדרגה עם נכחה על משך מדרגותיהם | ||
| + | |- | ||
| + | | | ||
| + | :*The first term of the "same", which is one, when it is composed with the first term of the "others", which is two, the first actual triangle is generated, which is three. <math>\scriptstyle{\color{blue}{1+2=3}}</math> | ||
| + | |style="text-align:right;"|כי המדרגה הראשונה מההוא הויים והוא האחד כאשר הורכבה עם המדרגה הראשונה מהזולתיים והוא ב' יחודש המשולש הראשון בפעל והוא ג‫' | ||
| + | |- | ||
| + | | | ||
| + | :*When the first term of the "others", which is two, is composed with the second term of the "same", which is four, the second actual triangle is generated, which is six. <math>\scriptstyle{\color{blue}{2+4=6}}</math> | ||
| + | |style="text-align:right;"|וכאשר תרכיב המדרגה הראשונה מהזולתיים והיא ב' עם המדרגה השנית מהוא הויים והוא ד' יחודש המשולש השני בפעל והוא ו‫' | ||
| + | |- | ||
| + | | | ||
| + | :So the combination of these terms is found always according to this description. | ||
| + | |style="text-align:right;"|וכן תמצא הרכבת אלו המדרגות בזה התאר תמיד | ||
| + | |- | ||
| + | | | ||
| + | :{|class="wikitable" style="color:blue; text-align:center;" | ||
| + | |- | ||
| + | |11||10||9||8||7||6||5||4||3||2 | ||
| + | |- | ||
| + | |21||19||17||15||13||11||9||7||5||3 | ||
| + | |- | ||
| + | |10||9||8||7||6||5||4||3||2||1 | ||
| + | |- | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | |יא||י||ט||ח||ז||ו||ה||ד||ג||ב | ||
| + | |- | ||
| + | |כא||יט||יז||טו||יג||יא||ט||ז||ה||ג | ||
| + | |- | ||
| + | |י||ט||ח||ז||ו||ה||ד||ג||ב||א | ||
| + | |} | ||
| + | |||
| + | |- | ||
| + | |It is visible from what was described and explained before, that when one of the dimensions of the square becomes different from the other by one, whether by addition to it or by subtraction from it, it becomes an "other". | ||
| + | |style="text-align:right;"|ויראה ממה שתארנו ובארנו קודם שהמרובע כאשר יתחלף באחד משני מרחקיו לאחד באחד אם בתוספת עליו ואם בחסרון ממנו יצא אל הזולתיי | ||
| + | |- | ||
| + | |Hence, the rank of the square that is equivalent to the "other" is as the rank of equality to the aforementioned five relations, since one of the two numbers exceeds the other and the other is less than it and the equality is a foundation for them. | ||
| + | |style="text-align:right;"|הנה אם כן מדרגת המרובע השוה לזולתיי כמדרגת השוה להקשות החמש אשר הקדמנו זכרם אחר שאחד משני המספרים נוסף על האחר והאחד חוסר ממנו והשווי פינה להם | ||
| + | |- | ||
| + | |It was also said that the true "same" is the one, and the true "other" is the duality. | ||
| + | |style="text-align:right;"|וכבר אמרנו גם כן שההוא הוא האמתי הוא האחד והזולתיי האמתי הוא השניות | ||
| + | |- | ||
| + | |In addition, the odd is similar to the "same" and the even to the "other", therefore, the regular square is similar to the "same", since it consists of the odds, and the [heteromecic number], whose two dimensions differ by one, is similar to the "other", since it consists of the evens. | ||
| + | |style="text-align:right;"|עוד אחר זה כבר ידמה הנפרד להוא הוא והזוג לזולת ולזה בעצמו כבר ידמה המרובע השוה ההוא הוא אחר שהוא מורכב מהנפרדים וידמה המתחלף שני המרחקים באחד הזולתיי אחר שהוא מורכב מהזוגות | ||
| + | |- | ||
| + | |Returning to what was mentioned about that which is evident concerning these two species, i.e. the "same" and the "other", when they are arranged successively as aforementioned, which is the succession of the two species together in one line, so that their terms are such that each term of one of the two species is between two terms of the other species successively. | ||
| + | |style="text-align:right;"|אמנם עתה נשוב אל מה שזכרנוהו ממה שיראה משני אלו המינים ר"ל ההוא והזולתיי כאשר יסודר כפי הסדר אשר זכרנוהו לפנים והוא סדר שני המינים יחד בטור אחד בסדר שיהיו מדרגותיהם כן כל מדרגה מאחד משני המינים בין שתי מדרגות מהמין האחד על המשך | ||
| + | |- | ||
| + | | | ||
| + | ::Such as: 1; 2; 4; 6; 9; and so on. | ||
| + | |style="text-align:right;"|כמו א' ב' ד' ו' ט' וכן תמיד | ||
| + | |- | ||
| + | |One finds that every three terms of this line, when it is arranged by this order, when they are equal in ratio, they differ in their additions to each other; and when they are equal in their additions to each other, they differ in their ratio. | ||
| + | |style="text-align:right;"|הנה זה הטור כאשר יסודר זה הסדר תמצא כל שלשה מדרגות מהם כאשר השתוו ביחס יתחלפו בהגעות תוספותיהם קצתם על קצת וכאשר ישתוו בהגעות תוספות קצתם על קצת יתחלפו ביחס | ||
| + | |- | ||
| + | | | ||
| + | :*1; 2; 4 - are in the double ratio [<math>\scriptstyle{\color{blue}{2:1=4:2}}</math>], but the excess of 4 over 2 is two [<math>\scriptstyle{\color{blue}{4-2=2}}</math>], and the excess of 2 over 1 is 1 [<math>\scriptstyle{\color{blue}{2-1=1}}</math>] - the ratio is one and the same, while the additions are different. | ||
| + | |style="text-align:right;"|כי א' ב' ד' ביחס הכפל השניי אבל תוספת ד' על ב' ב' ותוספת ב' על א' א' הנה היחס אחד והתוספות מתחלפות | ||
| + | |- | ||
| + | | | ||
| + | :*2; 4; 6 - the excess of 6 over 4 is the same as the excess of 4 over 2 [<math>\scriptstyle{\color{blue}{6-4=4-2}}</math>], but the ratio differs, as the ratio of 6 to 4 is not the ratio of 4 to 2 [<math>\scriptstyle{\color{blue}{6:4\ne4:2}}</math>]. | ||
| + | |style="text-align:right;"|אמנם שלשה המדרגות אשר הן ב' ד' ו' תוספת ו' על ד' כמו תוספת ד' על ב' אלא שהיחס יתחלף לפי שיחס ו' אל ד' בלתי יחס ד' אל ב‫' | ||
| + | |- | ||
| + | | | ||
| + | :*4; 6; 9 - are in one ratio, but their additions are unequal. | ||
| + | |style="text-align:right;"|וכן גם כן שלשת המדרגות אשר הן ד' ו' ט' שהן ביחס אחד ואין התוספות שוות | ||
| + | |- | ||
| + | | | ||
| + | :*6; 9; 12 - their additions [are equal], but they differ in their ratio. | ||
| + | |style="text-align:right;"|וכן גם כן שלשת מדרגות ו' ט' י"ב בהגעות התוספות מתחלפות ביחס | ||
| + | |- | ||
| + | | | ||
| + | :Likewise, every three terms of this line which is compounded from the "same" and the "others", are found according to the combination described in this description above. | ||
| + | |style="text-align:right;"|וכן תמצא כל שלשה מדרגות מזה הטור המורכב מן ההוא הויים והזולתיים כפי ההרכבה אשר תארנו לפנים בזה התאר | ||
| + | |- | ||
| + | |It is clarified from what was mentioned, that when the "other" and the "same" are such that the two dimensions of the "other" differ so that the length exceeds the breadth by one, for every three terms of this line that are matching in ratio, but differ in the excesses, the difference of the excesses is also one. | ||
| + | |style="text-align:right;"|ויתבאר ממה שזכרנו שכאשר היה הזולתיי וההוא הוא אלו ששני מרחקי הזולתיי יתחלפו בתוספת אחד באורך על הרחב שיהיו כל שלשה מדרגות מזה הטור שיסכימו ביחס ויתחלפו בהגעת התוספת שיהיה חלוף התוספות גם כן באחד | ||
| + | |- | ||
| + | | | ||
| + | :For, 2, which is the difference between 4 and 2 exceeds the one, which is the difference between 1 and 2, by one [<math>\scriptstyle{\color{blue}{\left(4-2\right)-\left(2-1\right)=2-1=1}}</math>], and so on. | ||
| + | |style="text-align:right;"|כי ב' אשר הוא המותר בין ד' וב' יוסיף על האחד אשר הוא המותר אשר הוא בין א' וב' אחד וכן תמיד | ||
| + | |- | ||
| + | |We further say what is strengthened by what was mentioned before, that the odds verify more strongly the nature of the "same", i.e. those that have roots: | ||
| + | |style="text-align:right;"|ונאמר עוד שממה שיחזק עם מה שזכרנוהו קודם כי הנפרדים יותר חזקי ההאמתה בטבע ההוא ר"ל הנגדרים מה שאנחנו זוכרים אותו | ||
| + | |- | ||
| + | | | ||
| + | :*Arranging the numbers that begin with the one, and proceeding by the double ratio, such as: 1; 2; 4; 8; 16; 32; 64. | ||
| + | |style="text-align:right;"|וזה שאנו נסדר המספרים אשר התחלתם מהאחד והמשכם ביחס הכפל השניי בטור כמו א' ב' ד' ח' י"ו ל"ב ס"ד | ||
| + | |- | ||
| + | | | ||
| + | :*Arranging also the numbers that begin with the one, and proceeding by the triple ratio, such as: 1; 3; 9; 27; 81; 243. | ||
| + | |style="text-align:right;"|ונסדר גם כן המספרים אשר התחלתם מהאחד והמשכם על יחס הכפל השלישיי כמו א' ג' ט' כ"ז פ"א רמ"ג | ||
| + | |- | ||
| + | |One finds that the odd terms in each of the two lines are of the "same", i.e. necessarily having root, i.e. the terms that are in a place whose number is odd, their values have roots; those that are in a place whose number is even, their values do not have roots. | ||
| + | |style="text-align:right;"|ונמצא מדרגות הנפרדים מכל אחד משני הטורים מספרים הוא הויים ר"ל שרשיים בהכרח ר"ל שהמדרגות אשר תהיינה במקום נפרד ממנין המדרגות הגעותיהם נגדרות ואשר הם במקום זוג ממנין המדרגות הגעותיהם בלתי נגדרות | ||
| + | |- | ||
| + | |It is further said that the solid cube numbers, since they are also similar to the "same", as their dimensions are equal, they are also produced from the odd numbers. | ||
| + | |style="text-align:right;"|ונאמר עוד שהמספרים הגרמיים המעוקבים אחר שהם גם כן דומים להוא הויים לפי שמרחקיהם שוים הנה הם יתילדו מהנפרדים גם כן | ||
| + | |- | ||
| + | |This is found by the natural wonderful technique that is easy to execute: | ||
| + | |style="text-align:right;"|וימצא זה בזאת התחבולה הנפלאה בטבע הנקלה במעשה | ||
| + | |- | ||
| + | | | ||
| + | :*Arranging the natural odds in a line. | ||
| + | |style="text-align:right;"|וזה שאנו נסדר הנפרדים הטבעיים בטור | ||
| + | |- | ||
| + | | | ||
| + | ::*The first potential cube, which is one, is the first potential odd | ||
| + | |style="text-align:right;"|וימצא המעוקב הראשון בכח אשר הוא האחד הוא הנפרד הראשון בכח אשר הוא א‫' | ||
| + | |- | ||
| + | | | ||
| + | ::*The second potential cube, which is eight, is from the combination of the two terms of the odds following the one, which are 3 and 5 <math>\scriptstyle{\color{blue}{8=3+5}}</math>. | ||
| + | |style="text-align:right;"|והמעוקב השני בכח הוא ח' מהרכבת שתי מדרגות ימשכו לאחד מהנפרדים והם ג' וה‫' | ||
| + | |- | ||
| + | | | ||
| + | ::*The third cube, which is 27, is from the combination of the three terms of the odds following the the two terms, from which the second cube is compounded, and they are 7, 9, 11, for their sum is 27. <math>\scriptstyle{\color{blue}{27=7+9+11}}</math>. | ||
| + | |style="text-align:right;"|והמעוקב השלישי אשר הוא כ"ז מהרכבת שלשה מדרגות ממדרגות הנפרדים ילוו לשתי המדרגות אשר הורכב מהם המעוקב השני והם ז' ט' י"א כי כל זה כ"ז | ||
| + | |- | ||
| + | |So, one always finds, whenever skipping to a cube, that its combination is from odd terms, whose number are the same as the number of its place among the terms of the cubes, i.e. that the second cube consists of two odds, the third consists of three odds, and so on for what follows. | ||
| + | |style="text-align:right;"|וכן תמצא זה תמיד כל מה שתדלג אל מעוקב תמצא כי הרכבתו ממדרגות נפרדים מנינם כמו מקומו ממנין מדרגות המעוקבים ר"ל כי המעוקב השני מהרכבת שני נפרדים והשלישי מהרכבת שלשה נפרדים וכן מה שאחר זה | ||
| + | |- | ||
| + | |Yet, for every cube, the first of the odds, from which it is composed, follows the end of the terms, from which its preceding cube is composed, and the terms are taken successively by the order of the natural odd numbers. | ||
| + | |style="text-align:right;"|אלא שכל מעוקב הנה ראש הנפרדים אשר מהם יורכב אחר סוף מדרגה מהמדרגות אשר הורכב מהם המעוקב אשר לפניו עוד המדרגות לקוחות על משך סדר הנפרדים הטבעיים | ||
| + | |- | ||
| + | |This is the rule of the discussion about it, and its diagram is as you see: | ||
| + | |style="text-align:right;"|הנה זה כלל המאמר על זה וצורתו כמו שתראה | ||
| + | |} | ||
| + | |||
| + | {|class="wikitable" style="margin-left: auto; margin-right: auto; text-align:center;" | ||
| + | |- | ||
| + | |מא||לט||לז||לה||לג||לא||כט||כז||כה||כג||כא||יט||יז||טו||יג||יא||ט||ז||ה||ג||א | ||
| + | |- | ||
| + | |colspan="6"|המעוקב הששי||colspan="5"|המעוקב החמישי||colspan="4"|המעוקב הרביעי||colspan="3"|המעוקב השלישי||colspan="2"|המעוקב השני||המעוקב הראשון | ||
| + | |- | ||
| + | |colspan="6"|ריו||colspan="5"|קכה||colspan="4"|סד||colspan="3"|כז||colspan="2"|ח||א | ||
| + | |} | ||
| + | {|class="wikitable" style="color:blue; margin-left: auto; margin-right: auto; text-align:center;" | ||
| + | |- | ||
| + | |41||39||37||35||33||31||29||27||25||23||21||19||17||15||13||11||9||7||5||3||1 | ||
| + | |- | ||
| + | |colspan="6"|sixth cube||colspan="5"|fifth cube||colspan="4"|fourth cube||colspan="3"|third cube||colspan="2"|second cube||first cube | ||
| + | |- | ||
| + | |colspan="6"|216||colspan="5"|125||colspan="4"|64||colspan="3"|27||colspan="2"|8||1 | ||
| + | |} | ||
| + | |||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | === [Relative Quantity] === | ||
| + | |||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | ==== The Discussion on The Ten Means ==== | ||
| + | |||
| + | |style="text-align:right;"|<big>הדבור על ה{{#annot:term|564,1741|Dn8O}}אמצעיים{{#annotend:Dn8O}} העשרה</big> | ||
| + | |- | ||
| + | |It is what the author of this book in Arabic calls al-ʽIyād. | ||
| + | |style="text-align:right;"|וזה אשר יקרא אותו בעל זה הספר בערבי אלעיאד | ||
| + | |- | ||
| + | |Its meaning: a determination of two proportions or more between two given terms, | ||
| + | |style="text-align:right;"|ומשמעותו הגבלת שני יחסים או יותר מזה בין גבולים מונחים | ||
| + | |- | ||
| + | | | ||
| + | *either by themselves, i.e. that the determination is actualized from the terms themselves to each other. | ||
| + | |style="text-align:right;"|אם מעצמם ר"ל שתהיה ההגבלה מוצאת מעצמי הגבולים עצמם קצת אל קצת | ||
| + | |- | ||
| + | | | ||
| + | *or by the excess of one over the other | ||
| + | |style="text-align:right;"|ואם מיתרון קצתם על קצת | ||
| + | |- | ||
| + | | | ||
| + | *or by both properties together. | ||
| + | |style="text-align:right;"|ואם משני העניינים יחד | ||
| + | |- | ||
| + | |The proportion is the being of two determined terms given with respect to each other. | ||
| + | |style="text-align:right;"|והיחס הוא ישות שני גבולים מוגבלים מונחים אחד מהם אצל האחר | ||
| + | |- | ||
| + | |This is at least a relation of three terms. | ||
| + | |style="text-align:right;"|ובפחות מה שיהיה ההתיחסות בשלשה גבולים | ||
| + | |- | ||
| + | | | ||
| + | :Such as: 4; 2; 1 | ||
| + | |style="text-align:right;"|כמו ד' וב' וא‫' | ||
| + | |- | ||
| + | | | ||
| + | :For the ratio of 4 to 2 is as the ratio of 2 to 1 <math>\scriptstyle{\color{blue}{2:4=1:2}}</math> | ||
| + | |style="text-align:right;"|כי שעור ד' אצל ב' כשעור ב' אצל א‫' | ||
| + | |- | ||
| + | | | ||
| + | :Also vice versa. The determination that is called al-ʽIyād, which is between 1 and 2 with respect to 4, their ratio is one [<math>\scriptstyle{\color{blue}{1:2=2:4}}</math>] | ||
| + | |style="text-align:right;"|וכמו כן בהפך הנה ההגבלה הנקראת אלאיעד אשר בין א' וב' אצל ד' הוא יחסם אחד | ||
| + | |- | ||
| + | |This al-ʽIyād is essentially the given number itself. | ||
| + | |style="text-align:right;"|וזה אלאיעד הוא בעצם המספר המונח עצמו | ||
| + | |- | ||
| + | |The numbers in this ratio can always be extended by [more] units, as explained before, more than once, in this book, so that the numbers will be four, or five, or six, or more, as one wishes. | ||
| + | |style="text-align:right;"|וזה היחס כבר אפשר שיתוספו מספריו תמיד באחדות כאשר קדם פירושינו לו יותר מפעם בספרנו זה כמו שיהיו המספרים כאשר היו ארבעה או חמשה או ששה או יותר מזה כפי מה שתרצה | ||
| + | |- | ||
| + | | | ||
| + | :Such as setting 1; 2; 4; 8; 16; 32; 64 - since all of them are in the ratio mentioned initially between the three terms. | ||
| + | |style="text-align:right;"|כמו שתניח א' ב' ד' ח' י"ו ל"ב ס"ד כי אלו כלם ביחס הנזכר בין שלשת הגבולים תחלה | ||
| + | |- | ||
| + | |The proportion that is subject to addition: | ||
| + | |style="text-align:right;"|ואמנם ההתיחס אשר יפול ביתרון | ||
| + | |- | ||
| + | | | ||
| + | :Such as: 1; 2; 3 | ||
| + | |style="text-align:right;"|כמו א' ב' ג‫' | ||
| + | |- | ||
| + | | | ||
| + | :For the measure of 1 to 2 is not as the measure of 2 to 3 [<math>\scriptstyle{\color{blue}{2:1\ne3:2}}</math>]. | ||
| + | |style="text-align:right;"|כי אין שעור האחד מב' כשעור ב' מג‫' | ||
| + | |- | ||
| + | | | ||
| + | :Yet, the measure of the excess of 3 over 2 is the measure of the excess of 2 over 1 [<math>\scriptstyle{\color{blue}{3-2=2-1}}</math>]; and vice versa. | ||
| + | |style="text-align:right;"|אבל שעור תוספת ג' על ב' הוא השעור אשר בו יוסיף ב' על האחד וכן הוא גם כן בהפך | ||
| + | |- | ||
| + | |It is al-ʽIyād even if the given numbers are numerous: | ||
| + | |style="text-align:right;"|וזה אלאיעד גם כן אם ירבו המספרים המונחים | ||
| + | |- | ||
| + | | | ||
| + | :The measures of the excesses according to this example are not equal also according to this example: 2; 3; 4; 5 [<math>\scriptstyle{\color{red}{4:2\ne5:3}}</math>]. | ||
| + | |style="text-align:right;"|הנה כפי זה המשל ימצאו שעורי התוספות בחלוף כפי זה המשל גם כן כמו ב' וג' וד' וה‫' | ||
| + | |- | ||
| + | | | ||
| + | :Yet the excess of 5 over 3 is as the excess of 4 over 2 [<math>\scriptstyle{\color{blue}{5-3=4-2}}</math>]; and vice versa. | ||
| + | |style="text-align:right;"|כי תוספת ה' על ג' כמו תוספת ד' על ב' וכן ימצא גם כן בהפך | ||
| + | |- | ||
| + | |It is said that the proportions that are acknowledged by all the ancients - '''Pythagoras''', '''Plato''' and others - are three: | ||
| + | |style="text-align:right;"|ונאמר שהאמצעיים אשר יודו בם כל הקדמונים ר"ל פיתאגורש ואפלאטון וזולתם הם שלשה | ||
| + | |- | ||
| + | | | ||
| + | :1) for arithmetic | ||
| + | |style="text-align:right;"|האחד מהם לחשבון | ||
| + | |- | ||
| + | | | ||
| + | :2) for geometry | ||
| + | |style="text-align:right;"|והשני למדות | ||
| + | |- | ||
| + | | | ||
| + | :3) for music | ||
| + | |style="text-align:right;"|והשלישי לחבור הנגונים | ||
| + | |- | ||
| + | | | ||
| + | :The geometric and the harmonic proportions have three subcontrary proportions: | ||
| + | |style="text-align:right;"|ולאמצעי המדות והחבור {{#annot:term|2515,2108|UErt}}מקבילים{{#annotend:UErt}} אחרים שלשה | ||
| + | |- | ||
| + | | | ||
| + | ::The harmonic proportion has one contrary | ||
| + | |style="text-align:right;"|לחבור הקבלה אחת | ||
| + | |- | ||
| + | | | ||
| + | ::The geometric proportion has two contraries | ||
| + | |style="text-align:right;"|ולמדות שתי הקבלות | ||
| + | |- | ||
| + | | | ||
| + | :These do not have names, they are called by their numbers: fourth, fifth, sixth. | ||
| + | |style="text-align:right;"|אלא שהם אינם בעלות שמות כמו אלו ונקראו מהמספר ונקראו רביעית וחמישית וששית | ||
| + | |- | ||
| + | |Four other proportions were added to these six proportions constituting the ten proportions, as the ten was perfect for Pytagoras. | ||
| + | |style="text-align:right;"|וכבר אמרו אחר זה המאמר הקדום שהאמצעיים עשרה והוסיפו על אלו הששה ארבעה אחרים להשלים האמצעיים העשרה להיות לעשרה שלמות אצל פיתאגורש | ||
| + | |- | ||
| + | |We will discuss the description of these proportions: | ||
| + | |style="text-align:right;"|ונדבר בתאר אלו האמצעיים | ||
| + | |- | ||
| + | | | ||
| + | ===== The Arithmetic Proportion ===== | ||
| + | |||
| + | |style="text-align:right;"|וניחד המאמר תחלה ב{{#annot:term|1769,2098|pyLZ}}מצוע המספריי{{#annotend:pyLZ}} | ||
| + | |- | ||
| + | |The discussion about the priority of the number in the four sciences is already completed. | ||
| + | |style="text-align:right;"|ונאמר הנה כבר נשלם מאמרנו בהיות המספר ראוי בקדימה באומניות הארבעה | ||
| + | |- | ||
| + | |The numbers given in the arithmetic proportion are according to the natural succession, or similarly to the natural succession. | ||
| + | |style="text-align:right;"|ועם זה הנה המספרים המונחים ל{{#annot:term|1769,1200|2N34}}אמצעי החשבון{{#annotend:2N34}} הם כפי סדר הטבע או מה שהיה דומה לסדר הטבע | ||
| + | |- | ||
| + | |Hence it is necessary to precede this proportion to the other proportions, since it is antecedent to the other antecedent proportions and all the more so to their opposites. | ||
| + | |style="text-align:right;"|הנה אם כן יחוייב בהכרח שנקדים זה {{#annot:term|564,1741|Tfg6}}האמצעי{{#annotend:Tfg6}} על שאר האמצעיים שאחר שהיה קודם על שאר האמצעיים הקודמים הנה יותר ראוי מזה שיקדם על המקבילים להם | ||
| + | |- | ||
| + | | | ||
| + | :{{#annot:definition|1769,2098|3uUu}}The arithmetic mean is when three numbers or more are given, set according to the natural addition successively. | ||
| + | |style="text-align:right;"|הנה האמצעי המספריי יהיה כאשר תניח שלשה מספרים או יותר מזה והונחו כפי חבור הטבע ימשכו קצתם לקצת{{#annotend:3uUu}} | ||
| + | |- | ||
| + | | | ||
| + | ::Such as: 1; 2; 3; 4; 5 | ||
| + | |style="text-align:right;"|כמו א' ב' ג' ד' ה‫' | ||
| + | |- | ||
| + | | | ||
| + | ::For, excess of each of these numbers over its preceding number is one. | ||
| + | |style="text-align:right;"|כי אלו המספרים בהכרח תוספת כל אחד מהם על מה שלפניו אחד | ||
| + | |- | ||
| + | |If one wishes to arrange them according to this ratio, which is equality of the difference: | ||
| + | |style="text-align:right;"|ואם תרצה לסדרם בזה היחס אשר הוא שווי היתרון | ||
| + | |- | ||
| + | | | ||
| + | :*Setting the natural numbers in a line. | ||
| + | |style="text-align:right;"|אתה תסדר המספרים הטבעיים בטור | ||
| + | |- | ||
| + | | | ||
| + | :*Starting with the number that is wished to be set as the first term, then examining how many ranks [units], according to the natural succession, are between it and the number that is wished to be set as the mean term, so that between the mean and the third term will succeed the same number of ranks [units], and also between the third and the fourth, or whichever terms that are set in this proportion. | ||
| + | |style="text-align:right;"|עוד תתחיל במספר אשר תרצה לשומו בגבול הראשון ותעיין כמה בינו ובין המספר אשר תרצה לשומו {{#annot:term|2121,1741|POeO}}אמצעי{{#annotend:POeO}} מהמדרגות אשר על סדר הטבע וימשכו כמו אותו המנין מהמדרגות בין האמצעי והגבול השלישי וכן בין השלישי והרביעי או במה שתניח מהמדרגות בזה היחס | ||
| + | |- | ||
| + | | | ||
| + | ::Such as: setting the first term as 2 and the second term as 4. | ||
| + | |style="text-align:right;"|כמו שתניח הגבול הראשון ב' והגבול השני ד‫' | ||
| + | |- | ||
| + | | | ||
| + | ::No doubt that the third term will be set according to the distance from 4 to 2, which is the distance from 6 to 4, i.e. a distance of two ranks [units]. | ||
| + | |style="text-align:right;"|ואין ספק שתניח הגבול השלישי על דמיון מרחק ד' מב' והוא מרחק ו' מד' ר"ל על מרחק שתי מדרגות | ||
| + | |- | ||
| + | | | ||
| + | ::Also, when setting a fourth term, it is the one that has distance from 6 of two ranks, which is 8. | ||
| + | |style="text-align:right;"|וכן אם תניח גבול רביעי היה הוא אשר ירחק מו' בשתי מדרגות והוא ח‫' | ||
| + | |- | ||
| + | |According to this way the terms are set. When you wish to increase them, their excess is always by the same addition. | ||
| + | |style="text-align:right;"|וכפי זה הדרך תניח הגבולים כאשר תרצה להרבותם הנה תהיה הוספתם לעולם בתוספת אחד | ||
| + | |- | ||
| + | | | ||
| + | *The property of this proportion, i.e. the arithmetic proportion, that is necessary for it and for no other: its mean term, when it is doubled, if the given terms have one mean, equals the sum of the two extremes, | ||
| + | :<math>\scriptstyle2a_2=a_1+a_3</math> | ||
| + | |style="text-align:right;"|ובפרט זה האמצעי ר"ל אמצעי החשבון המתחייב לאלו בלתי זולתם שהגבול האמצעי מהם כשיכפל על ב' אם היו המדרגות המונחות להם אמצעי אחד ישוה שני הקצוות כאשר יקובצו | ||
| + | |- | ||
| + | | | ||
| + | :and if the terms have two mean terms, the sum of the two means equals the sum of the two extremes: | ||
| + | :<math>\scriptstyle a_2+a_3=a_1+a_4</math> | ||
| + | |style="text-align:right;"|אם היו המדרגות בעלות שני אמצעיים הנה קבוץ שני האמצעים כמו קבוץ שני הקצוות | ||
| + | |- | ||
| + | | | ||
| + | *This proportion has a second property: the ratio of each term to itself is equal to the ratio of the differences of the terms to each other. | ||
| + | :<math>\scriptstyle a_i:a_i=\left(a_{n+1}-a_n\right):\left(a_{m+1}-a_m\right)</math> | ||
| + | |style="text-align:right;"|ולהם סגולה שנית גם כן והיא שיחס כל אחד אל עצמו כמו יחס מותרי הגבולים אלו על אלו קצתם על קצת | ||
| + | |- | ||
| + | | | ||
| + | *Also a third property, which is more difficult and hidden from the understanding of many: the product of the two extremes one by the other compared to the product of the mean [by itself] is smaller by the product of the differences of the terms by each other, whether the terms are odd in number, or whichever number they are, so that when they are summed they are more than the two extremes together. | ||
| + | ::<math>\scriptstyle\left(a_2\right)^2-\left(a_1\sdot a_3\right)=\left(a_2-a_1\right)\sdot\left(a_3-a_2\right)</math> | ||
| + | |style="text-align:right;"|עוד סגולה שלישית והיא היותר קשה ונעלמת מדעת רבים והיא כי כפילת שתי הקצוות האחד על האחר כאשר נערכהו אל כפילת האמצעי הוא יותר פחות בערך כפילת מותרי הגבולים אלו על אלו בין שיהיו הגבולים נפרדים במספרם ובין שיהיו איזה מספר שיהיה כאשר יקובצו היו יותר משני הקצוות יחד | ||
| + | |- | ||
| + | | | ||
| + | *This proportion has a fourth property, mentioned by the ancients: the ratio between the smaller terms is larger than the ratio between the greater terms | ||
| + | |style="text-align:right;"|ולהם סגולה רביעית והיא ממה שזכרנוהו הראשונים מתאריהם והיא שיחס אשר בין הגבולים הקטנים מהם יותר גדול מיחס אשר בין הגבולים הגדולים | ||
| + | |- | ||
| + | | | ||
| + | ::Such as: 2; 3; 4 - which are in this proportion. | ||
| + | |style="text-align:right;"|כי שנים ושלשה וארבעה אשר הם בסדר זה האמצעי | ||
| + | |- | ||
| + | | | ||
| + | ::The ratio of 4, which is the greater term, to 3 is as the sesquitertian ratio <math>\scriptstyle{\color{blue}{4:3=1+\frac{1}{3}}}</math> | ||
| + | |style="text-align:right;"|שעור ארבעה מהם והוא הגבול הגדול אצל השלשה כיחס המוסיף חלק השלישי | ||
| + | |- | ||
| + | | | ||
| + | ::The measure of 3 to 2, which is the smaller term, is as the sesquialter ratio <math>\scriptstyle{\color{blue}{3:2=1+\frac{1}{2}}}</math> | ||
| + | |style="text-align:right;"|ושעור שלשה אצל השנים והוא הגבול הקטן כיחס המוסיף חלק החציי | ||
| + | |- | ||
| + | | | ||
| + | ::This ratio [= the sesquialter] is greater than the first [= the sesquitertian] as the measure of the excess of the half over the third. | ||
| + | |style="text-align:right;"|וזה היחס גדול מהראשון כשעור תוספת החצי על השליש | ||
| + | |- | ||
| + | | | ||
| + | :The opposite is seen in the harmonic proportion, in which the ratio between the greater terms is larger than the ratio between the smaller [terms]. | ||
| + | |style="text-align:right;"|וזה יראה ביחס הניגוניי בהפך כי היחס אשר בין הגבולים הגדולים יותר גדול מהיחס אשר הקטנים | ||
| + | |- | ||
| + | | | ||
| + | :Because of this, the arithmetic proportion is subcontrary to the harmonic proportion, | ||
| + | |style="text-align:right;"|מפני זה {{#annot:term|1769,1174|Hioi}}אמצעי המספר{{#annotend:Hioi}} הם הפכים ל{{#annot:term|1771,2394|A06f}}אמצעי הנגון{{#annotend:A06f}} | ||
| + | |- | ||
| + | | | ||
| + | :and the geometric proportion is mean between these opposites, for [in this proportion] the ratio between the greater terms is equal to the ratio between the smaller [terms] | ||
| + | |style="text-align:right;"|ואמצעי המדות הם אמצעיי בין אלו ההפכים כי יחס אשר בין הגבולים הגדולים הוא שוה ליחס אשר בין הקטנים | ||
| + | |- | ||
| + | |This is the end of the discussion of the arithmetic proportion. | ||
| + | |style="text-align:right;"|זה הוא סוף המאמר על {{#annot:term|1769,2098|hwlm}}האמצעי המספרי{{#annotend:hwlm}} | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ===== The Geometic Proportion ===== | ||
| + | |||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :{{#annot:definition|1770,1744|o5gn}}The geometic proportion is when there are three numbers or more, such that the measure of the first of them to the second is as the measure of the second to the third and vice versa. | ||
| + | :<math>\scriptstyle a_1:a_2=a_2:a_3\longleftrightarrow a_2:a_1=a_3-a_2</math> | ||
| + | |style="text-align:right;"|ואמנם האמצעי המדותיי הוא כאשר יהיו שלשה מספרים או יותר מזה והיה שעור הראשון מהם אצל השני כשעור השני אצל השלישי וכן בהפך{{#annotend:o5gn}} | ||
| + | |- | ||
| + | | | ||
| + | ::Such as: 4; 8; 16 - which are by this description in the geometic proportion. | ||
| + | |style="text-align:right;"|כמו ד' ח' י"ו שהם בזה התאר ב{{#annot:term|1770,1744|ppSt}}התיחסות המדותיי{{#annotend:ppSt}} | ||
| + | |- | ||
| + | |These given numbers in the geometric proportion differ from the given numbers in the arithmetic proportion, since these terms themselves are proportional and their differences between each other are unequal, while the differences of the former are equal and their terms are disproportional. | ||
| + | |style="text-align:right;"|וכבר יתחלפו אלו המספריים המונחים ל{{#annot:term|1770,1644|9OI3}}אמצעי ההנדסה{{#annotend:9OI3}} למספרים המונחים באמצעי המספר לפי שאלו המדרגות עצמן מתיחסות ומותרי קצתם על קצת בלתי שוה ואותן היו מותריהם שוים ומדרגותיהם בלתי מתיחסות | ||
| + | |- | ||
| + | | | ||
| + | *The property of the geometric proportion is that the ratio of the excesses of the terms one over the other is the same as the ratio of the terms themselves. | ||
| + | :<math>\scriptstyle\left(a_3-a_2\right):\left(a_2-a_1\right)=a_3:a_2=a_2:a_1</math> | ||
| + | |style="text-align:right;"|וסגלת זה האמצעי ההנדסיי כי למותרי הגבולים קצתם על קצת מהיחס כמו מה של גבולים עצמם מהיחס | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(16-8\right):\left(8-4\right)=8:4=16:8=8:4}}</math> | ||
| + | |style="text-align:right;"|כי יחס מותרי י"ו על ח' והוא ח' אל מותרי ח' על ד' והוא ד' כמו יחס י"ו אל ח' וח' אל ד‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Also if the terms are in triple ratio, such as: 3; 9; 27 | ||
| + | |style="text-align:right;"|וכן אם היו גם כן הגבולים ביחס הכפל השלישיי כמו ג' ט' כ"ז | ||
| + | |- | ||
| + | | | ||
| + | :Likewise always, for any given terms in whichever of the five ratios that one wishes, since the terms following according to the ratio - understand this. | ||
| + | |style="text-align:right;"|וכן הוא תמיד כל מה שתניח מן המדרגות באי זה מן היחסים החמש שתרצה אחר שיהיו הגבולים נמשכים ביחס והבן זה | ||
| + | |- | ||
| + | | | ||
| + | *This proportion has a second property: the product of the mean term by itself is as the two extremes multiplied one of them by the other. | ||
| + | :<math>\scriptstyle\left(a_2\right)^2=a_1\sdot a_3</math> | ||
| + | |style="text-align:right;"|ולזה האמצעי סגולה שנית והוא שמוכה הגבול האמצעי בעצמו כמו שני הקצוות מוכה אחד מהם באחר | ||
| + | |- | ||
| + | |It should be noticed to what was said before, concerning the "same" and "other" numbers, that between each term of one of them there is a mean term of the other successively in the natural order. | ||
| + | |style="text-align:right;"|וראוי שנשים לב למה שאמרנו לפנים שמספרי ההוא הוא והזולת בין כל מדרגה מאחד מהם ומדרגה מן האחר אמצעי על המשכם בסדר הטבע | ||
| + | |- | ||
| + | | | ||
| + | ::Such as: 4; 6; 9; 12; 16 | ||
| + | |style="text-align:right;"|כמו ד' ו' ט' י"ב י"ו | ||
| + | |- | ||
| + | |Since every three [subsequent] terms among them [starting with as "same" number] are proportional. | ||
| + | |style="text-align:right;"|כי כל שלשה מספרים יוקחו מהם מתיחסים | ||
| + | |- | ||
| + | |If their first term is "other" the differences are equal. | ||
| + | |style="text-align:right;"|ואם יהיה הגבול הראשון מהם זולתיי יהיה שווי היתרון | ||
| + | |- | ||
| + | |The rule of this statement: between every two subsequent "same" numbers there is one "other" number that follows the same ratio with them, which is less[?] than for the cube "same" numbers. | ||
| + | |style="text-align:right;"|וכלל זה המאמר שיפל בין כל שני מספרים הוא הויים נלוים מספר אחד זולתיי ימשך עמם על יחס בפחות מההוא הויים המעוקבים | ||
| + | |- | ||
| + | |Since between every two subsequent cubes among them there are two numbers that follow the same ratio. | ||
| + | |style="text-align:right;"|כי בין כל שני מעוקבים נלוים מהם שני מספרים ימשכו עמם על יחס | ||
| + | |- | ||
| + | | | ||
| + | ::Because the first actual cube is 8, the second cube is 27, and between these two cube numbers there are two numbers, which are 18 and 12, and these four are in the same ratio. | ||
| + | |style="text-align:right;"|כי המעוקב הראשון בפעל ח' והמעוקב השני כ"ז ובין שני אלו המספרים המעוקבים שני מספרים והם י"ח וי"ב וכבר יתיחסו אלו הארבעה ביחס אחד | ||
| + | |- | ||
| + | |This is what was necessary to be said here. | ||
| + | |style="text-align:right;"|וזה ממה שיחוייב שנאמר אותו הנה | ||
| + | |- | ||
| + | | | ||
| + | *square × square = square | ||
| + | |style="text-align:right;"|דע שכל מספר מרובע הוכה במספר מרובע הנה המקובץ מרובע | ||
| + | |- | ||
| + | | | ||
| + | *[cube] × [cube] = cube | ||
| + | |style="text-align:right;"|וכל מספר מרובע יוכה במספר בלתי מרובע יהיה המתקבץ מספר מעוקב | ||
| + | |- | ||
| + | | | ||
| + | ::Such as: <math>\scriptstyle{\color{blue}{8\sdot27=216=6\sdot6\sdot6}}</math> | ||
| + | |style="text-align:right;"|כמו ח' בכ"ז שהוא רי"ו כי זה מהכאות בו' עוד בו‫' | ||
| + | |- | ||
| + | | | ||
| + | *cube × "other" ≠ cube | ||
| + | |style="text-align:right;"|ואם תכה מעוקב בזולתיי לא יתקבץ מעוקב | ||
| + | |- | ||
| + | | | ||
| + | ::Such as: <math>\scriptstyle{\color{blue}{8\sdot6=48}}</math>, 48 is not a cube and not a rooted surface. | ||
| + | |style="text-align:right;"|כמו ח' בו' שהוא מ"ח ומ"ח אינו מעוקב ולא שטח נגדר | ||
| + | |- | ||
| + | | | ||
| + | *"other" × "other" = not necessarily a square or a cube | ||
| + | |style="text-align:right;"|ואם תכה מספר זולתיי במספר אחר זולתיי לא יחוייב שיתקבץ מרובע פשוט ולא גם כן מעוקב | ||
| + | |- | ||
| + | | | ||
| + | *even × even = even | ||
| + | |style="text-align:right;"|ואם תכה זוג בזוג יהיה המתקבץ זוג | ||
| + | |- | ||
| + | | | ||
| + | *odd × odd = odd | ||
| + | |style="text-align:right;"|ואם תכה נפרד בנפרד יהיה מה שיתקבץ נפרד | ||
| + | |- | ||
| + | |'''The author of this book''' [= Nicomachus] '''said''': '''Plato''' has already explained it in his book that is called in Greek language politeia. | ||
| + | |style="text-align:right;"|<big>אמר מניח הספר</big> וכבר באר זה אפלאטון בספרו אשר יכונה בלשון יוני בולוטיא | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ===== The Harmonic Proportion ===== | ||
| + | | | ||
| + | |- | ||
| + | |{{#annot:definition|1771,2095|XLBu}}The harmonic proportion is when there are three given numbers, which are not in one ratio to each other, as the terms in the geometric proportion, and the excesses of each over the other are not equal, as [those of] the terms in the arithmetic proportion, but the measure of the greater term to the measure of the smaller term is as the measure of the excess of the greater term over the mean term to the excess of the mean over the smaller. | ||
| + | :<math>\scriptstyle a_3:a_1=\left(a_3-a_2\right):\left(a_2-a_1\right)</math> | ||
| + | |style="text-align:right;"|ואמנם האמצעי החבוריי הנה הוא כאשר היו שלשה מספרים מונחים ולא יהיה לקצתם אל קצת יחס אחד כמו הגבולים המדותיים לא יתרוני קצתם על קצת שוים כמו הגבולים המספרים אבל יהיה השעור הגבול הגדול אצל שעור הגבול הקטן כשעור מותר הגבול הגדול על הגבול האמצעי אל מותר האמצעי על הקטן{{#annotend:XLBu}} | ||
| + | |- | ||
| + | | | ||
| + | ::Such as: 3; 4; 6 | ||
| + | |style="text-align:right;"|כמו ג' ד' ו‫' | ||
| + | |- | ||
| + | |Also, the measure of the greater term to the greater excess is as the measure of the smaller term to the smaller excess. | ||
| + | |style="text-align:right;"|וכן גם כן שעור הגבול הגדול אצל גדול שני המותרים כשעור הגבול הקטן אצל קטן שני המותרים | ||
| + | |- | ||
| + | | | ||
| + | *The property of these terms is opposite to the terms of the arithmetic and the geometric proportion together. | ||
| + | |style="text-align:right;"|וסגולת אלו הגבולים הפכית לגבולי אמצעי החשבון והמדות יחד | ||
| + | |- | ||
| + | | | ||
| + | :*Since the ratio of the smaller of these terms to the mean is greater than the ratio of the mean to the greater. | ||
| + | ::[<math>\scriptstyle a_1:a_2>a_2:a_3</math>] | ||
| + | |style="text-align:right;"|לפי שאלו הגבולים יחס הגבול הקטן מהם אל האמצעי גדול מיחס האמצעי אל הגדול | ||
| + | |- | ||
| + | | | ||
| + | :*While in the terms of the arithmetic proportion, the ratio of the smaller to the mean is less than the ratio of the mean to the greater. | ||
| + | ::[<math>\scriptstyle a_1:a_2<a_2:a_3</math>] | ||
| + | |style="text-align:right;"|וגבולי האמצעי החשבון יחס הקטן אל האמצעי פחות מיחס האמצעי אל הגדול | ||
| + | |- | ||
| + | | | ||
| + | :*And in the terms of the geometric proportion, if three terms of them are given according to this condition, all of them are in one ratio. | ||
| + | ::[<math>\scriptstyle a_1:a_2=a_2:a_3</math>] | ||
| + | |style="text-align:right;"|ואמנם גבולי {{#annot:term|1770,2275|YtZ7}}אמצעי המדות{{#annotend:YtZ7}} אם היו שלשה גבולים מהם מונחים כפי תנאם יהיו כלם ביחס אחד | ||
| + | |- | ||
| + | | | ||
| + | *The harmonic proportion has another property, which is that the sum of the products of each of the two extreme terms by the mean is twice the product of the two extremes multiplied by each other. | ||
| + | :<math>\scriptstyle\left(a_1\sdot a_2\right)+\left(a_3\sdot a_2\right)=2\sdot\left(a_1\sdot a_3\right)</math> | ||
| + | |style="text-align:right;"|ולאמצעי חבוריי סגלה אחרת והיא שהכאת כל אחד משני הקצוות מגבוליו באמצעי מקובצים כמו כפל מה שיהיה משני הקצוות מוכה אחד מהם באחר | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ===== [Extracting a Third Term in the Arithmetic, Geometric, and Harmonic Proportions] ===== | ||
| + | |||
| + | | | ||
| + | |- | ||
| + | |Now it will be explained how the third term is extracted for each of these three proportions, when two of the terms are given: | ||
| + | |style="text-align:right;"|ונבאר עתה איך יוצא הגבול השלישי לכל אחד מאלו השלשה אמצעיים כאשר היו שני חלקים מהם מונחים | ||
| + | |- | ||
| + | | | ||
| + | *If two successive terms of the arithmetic proportion are given and the third term is required: | ||
| + | |style="text-align:right;"|וזה שאם היו שני חלקים מאמצעי החשבון נלוים מונחים ונבקש הגבול השלישי | ||
| + | |- | ||
| + | | | ||
| + | :*If the required is the greater term | ||
| + | ::*It is defined as a number such that the measure of its excess over the mean is as the measure of the excess of the mean over the smaller. | ||
| + | :::<math>\scriptstyle X-a_2=a_2-a_1</math> | ||
| + | |style="text-align:right;"|הנה אנו נשימהו אם היה הדרוש הגבול הגדול מספר ששעור תוספתו על האמצעי כשעור תוספת האמצעי על הקטן | ||
| + | |- | ||
| + | | | ||
| + | ::*The third term is found also, when it is the greater, by multiplying the mean, then subtracting from it the smaller term, and the remainder is the greater term. | ||
| + | :::<math>\scriptstyle a_3=X=2a_2-a_1</math> | ||
| + | |style="text-align:right;"|ונמצא גם כן הגבול השלישי כאשר היה הגדול כאשר נכפול האמצעי עוד נשליך ממנו הגבול הקטן ומה שישאר הוא הגבול הגדול | ||
| + | |- | ||
| + | | | ||
| + | :It was already said that the arithmetic proportion is that, whose terms exceed each other equally, but the terms are not proportional. | ||
| + | |style="text-align:right;"|הנה כבר נאמר שאמצעי החשבון הוא אשר יוסיפו גבוליו קצתם על קצת בשוה ואינם מתיחסים הגבולים | ||
| + | |- | ||
| + | | | ||
| + | :Furthermore, it was said that double the mean is as the two extremes together. | ||
| + | :<math>\scriptstyle2a_2=a_1+a_3</math> | ||
| + | |style="text-align:right;"|ואמרנו שכפל האמצעי מגבוליו כמו שני קצוותיו יחד | ||
| + | |- | ||
| + | | | ||
| + | :*If the required is the smaller term | ||
| + | ::*It is defined similarly as a number such that its subtraction from the mean is as the subtraction of the mean from the greater. | ||
| + | :::<math>\scriptstyle a_2-X=a_3-a_2</math> | ||
| + | |style="text-align:right;"|וכדמיון זה נוציא הגבול הקטן אם היה הדרוש וזה שאנו נשים הגבול הקטן מספר יהיה חסרונו מהאמצעי כשעור חסרון האמצעי מהגדול | ||
| + | |- | ||
| + | | | ||
| + | ::*Or, subtracting the greater term from double the mean - the remainder is the smaller term. | ||
| + | :::<math>\scriptstyle a_1=X=2a_2-a_3</math> | ||
| + | |style="text-align:right;"|או כפל האמצעי הנה נשליך ממנו הגבול הגדול ומה שישאר הוא הגבול הקטן | ||
| + | |- | ||
| + | | | ||
| + | :*If the required is the mean | ||
| + | |style="text-align:right;"|אמנם אם היה הדרוש באמצעי | ||
| + | |- | ||
| + | | | ||
| + | ::*Taking half the sum of the two extremes - it is the mean. | ||
| + | :::<math>\scriptstyle a_2=X=\frac{1}{2}\sdot\left(a_1+a_3\right)</math> | ||
| + | |style="text-align:right;"|אנו נקח חצי שני הקצוות מקובצים והוא האמצעי | ||
| + | |- | ||
| + | | | ||
| + | *Extracting the third term in the geometric proportion: | ||
| + | |style="text-align:right;"|ואמנם איך יוצא הגבול השלישי אל אמצעי המדות | ||
| + | |- | ||
| + | | | ||
| + | :*If the required is the greater term | ||
| + | |style="text-align:right;"|הנה אם היה דרושנו הגבול הגדול | ||
| + | |- | ||
| + | | | ||
| + | ::*It is defined as a number such that the ratio of the mean to it is as the ratio of the smaller to the mean. | ||
| + | :::<math>\scriptstyle a_2:X=a_1:a_2</math> | ||
| + | |style="text-align:right;"|אנו נשימהו מספר יחס האמצעי אליו כיחס הקטן אל האמצעי | ||
| + | |- | ||
| + | | | ||
| + | ::*Or, multiplying the mean by itself, then dividing the product by the smaller term - the result of division is the greater term. | ||
| + | :::<math>\scriptstyle a_3=X=\frac{\left(a_2\right)^2}{a_1}</math> | ||
| + | |style="text-align:right;"|או נכה האמצעי בעצמו ומה שיצא נחלקהו על הגבול הקטן ומה שיצא בחלוק הוא הגבול הגדול | ||
| + | |- | ||
| + | | | ||
| + | :It was already explained before that the geometric proportion is that, whose terms are proportional, but their excesses are unequal. | ||
| + | |style="text-align:right;"|וכבר בארנו לפנים שאמצעי המדות הוא אשר גבוליו מתיחסים ואין תוספתם שוה | ||
| + | |- | ||
| + | | | ||
| + | :It was further explained that the product of the mean by itself is as the product of the two extremes multiplied by each the other. | ||
| + | :<math>\scriptstyle\left(a_2\right)^2=a_1\sdot a_3</math> | ||
| + | |style="text-align:right;"|ובארנו הכאת שהאמצעי בעצמו כמו שני הקצוות מוכה אחד מהם באחר | ||
| + | |- | ||
| + | | | ||
| + | :For the number that is the quotient resulting from the division of that number [i.e. the square of the mean] by one of the two numbers that are multiplied one by the other [i.e. the upper and lower terms], is equal to the one of them, by which it is not divided. | ||
| + | :[<math>\scriptstyle\frac{\left(a_2\right)^2}{a_1}=a_3;\;\frac{\left(a_2\right)^2}{a_3}=a_1</math>] | ||
| + | |style="text-align:right;"|כי המספר אשר יצא מחלוקת אותו המספר על אחד משני המספרים המוכה אחד מהם באחר שוה לאשר לא יחלק עליו מהם | ||
| + | |- | ||
| + | | | ||
| + | :*If the required is the mean term | ||
| + | |style="text-align:right;"|ואם היה הדרוש הגבול האמצעי | ||
| + | |- | ||
| + | | | ||
| + | ::*Extracting the root of the product of one of the numbers by the other is defined as the mean. | ||
| + | :::<math>\scriptstyle a_2=X=\sqrt{a_1\sdot a_3}</math> | ||
| + | |style="text-align:right;"|הנה אנו נקח שורש המתקבץ מהכאת אחד משני המספרים באחר ונשימהו האמצעי | ||
| + | |- | ||
| + | | | ||
| + | *Extracting the third term in the harmonic proportion | ||
| + | |style="text-align:right;"|ואמנם איך נוציא הגבול השלישי מ{{#annot:term|1771,1208|UZha}}אמצעי החבור{{#annotend:UZha}} | ||
| + | |- | ||
| + | | | ||
| + | :*It is impossible to extract one of the two extreme terms. | ||
| + | |style="text-align:right;"|הנה אין אחד משני גבולי הקצוות אפשרי להוציאו | ||
| + | |- | ||
| + | | | ||
| + | ::This is because the extraction of each of them requires knowing two unknowns of the four proportional dividends in the ratio: | ||
| + | |style="text-align:right;"|וזה שיצטרך בהוצאת כל אחד מהם אל שתי ידיעות מוסכלות מארבעה מתיחסים חלוקים ביחס | ||
| + | |- | ||
| + | | | ||
| + | ::*One of them is the unknown term itself. | ||
| + | |style="text-align:right;"|אחד מהם בגבול המוסכל עצמו | ||
| + | |- | ||
| + | | | ||
| + | ::*The other is the excess of the unknown term over the mean term, if the unknown term is the greater term. | ||
| + | |style="text-align:right;"|והשני מותר הגבול המוסכל על הגבול האמצעי אם היה הגבול המוסכל הוא הגבול הגדול | ||
| + | |- | ||
| + | | | ||
| + | :::Or the excess of the mean over the smaller, if the unknown is the smaller. | ||
| + | |style="text-align:right;"|או מותר האמצעי על הקטן אם היה המוסכל הוא הקטן | ||
| + | |- | ||
| + | | | ||
| + | :*Extracting the mean term | ||
| + | |style="text-align:right;"|ואמנם הוצאת האמצעי | ||
| + | |- | ||
| + | | | ||
| + | ::*It is already known that this is when the excess of the greater over the smaller is taken and divided into two parts, such that the ratio of one of them to the other is as the ratio of the greater to the smaller. | ||
| + | |style="text-align:right;"|הנה כבר נודע שהוא כאשר לוקח מותר הגדול על הקטן וחולק בשני חלקים יחס א' מהם אל האחר יחס הגדול אל הקטון | ||
| + | |- | ||
| + | | | ||
| + | :::For, the smaller of the two parts of the excess, when it is added to the smaller of the two extremes, the result is the mean, and when the greater part of the two parts of the excess is subtracted from the greater term, it is also the mean. | ||
| + | |style="text-align:right;"|כי הקטון משני חלקי המותר כאשר נוסף על הקטן משני הקצוות היה המתקבץ הוא האמצעי וכאשר חוסר החלק הגדול משני חלקי המותר מהגבול הגדול היה גם כן הוא האמצעי | ||
| + | |- | ||
| + | | | ||
| + | :*Another way is by multiplication: multiplying one of the two extremes by the other, doubling the product, then dividing the result by the sum of the two extremes - the quotient is the mean. | ||
| + | :::<math>\scriptstyle a_2=X=\frac{2\left(a_1\sdot a_3\right)}{a_1+a_3}</math> | ||
| + | |style="text-align:right;"|ואמנם אופן אחר ההכאה בם אנו נכה אחד משני הקצוות באחר ועוד נכפל מה שיתקבץ ונחלק מה שיתקבץ על שני הקצוות מקובצים ומה שיצא מהחלוקה הוא האמצעי | ||
| + | |- | ||
| + | |This is enough for the description of the three proportions that are recognized by the ancients. | ||
| + | |style="text-align:right;"|וזה מספיק בתאר האמצעים השלשה המפורסמים אצל הקדמונים | ||
| + | |- | ||
| + | |The discussion on the remaining will be brief, as the ancients rarely used them. | ||
| + | |style="text-align:right;"|ואמנם הנשארים נקצר המאמר עליהם למעוט השתמש הקדמונים מהם | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ===== The Fourth Proportion ===== | ||
| + | |||
| + | |style="text-align:right;"|האמצעי הרביעי | ||
| + | |- | ||
| + | | | ||
| + | :It is the first of the seven remaining proportions. | ||
| + | |style="text-align:right;"|הוא הראשון מהשבעה האמצעים הנשארים | ||
| + | |- | ||
| + | | | ||
| + | :It is the one that is said to be opposite to the harmonic proportion. | ||
| + | |style="text-align:right;"|והוא אשר יאמר לו {{#annot:term|2515,2108|POnR}}מקביל{{#annotend:POnR}} {{#annot:term|1771,2095|ZR1k}}האמצעי החבוריי{{#annotend:ZR1k}} | ||
| + | |- | ||
| + | |When there are three terms, the ratio of the excess of the mean over the smaller to the excess of the greater over the mean is as the ratio of the greater to the smaller. | ||
| + | :<math>\scriptstyle\left(a_2-a_1\right):\left(a_3-a_2\right)=a_3:a_1</math> | ||
| + | |style="text-align:right;"|והוא שיהיו שלשה גבולים יחס מותר האמצעי על הקטן אל מותר הגדול על האמצעי כיחס הגדול אל הקטן | ||
| + | |- | ||
| + | | | ||
| + | ::Such as: 3; 5; 6 | ||
| + | |style="text-align:right;"|כמו ג' ה' ו‫' | ||
| + | |- | ||
| + | | | ||
| + | *Its property: the product of the greater by the mean is double the smaller by the mean. | ||
| + | :[the general property <math>\scriptstyle a_3\sdot a_2=2\sdot\left(a_1\sdot a_2\right)</math> is incorrect] | ||
| + | |style="text-align:right;"|וסגולת זה האמצעי הוא שהכאת הגדול באמצעי כפל הקטן באמצעי | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ===== The Fifth Proportion ===== | ||
| + | |||
| + | |style="text-align:right;"|והאמצעי החמשי | ||
| + | |- | ||
| + | | | ||
| + | :One of the two proportions that are opposite to the geometrical proportion. | ||
| + | |style="text-align:right;"|והוא אחד משני המקבילים ל{{#annot:term|1770,1744|2w0R}}אמצעי המדותיי{{#annotend:2w0R}} | ||
| + | |- | ||
| + | |When there are three terms, the ratio of the excess of the greater over the mean to the excess of the mean over the smaller is as the ratio of the smaller to the mean. | ||
| + | :<math>\scriptstyle\left(a_3-a_2\right):\left(a_2-a_1\right)=a_1:a_2</math> | ||
| + | |style="text-align:right;"|הוא כאשר יהיו שלשה גבולים יחס מותר הגדול על האמצעי אל מותר האמצעי על הקטן כיחס הקטן אל האמצעי | ||
| + | |- | ||
| + | | | ||
| + | ::Such as: 2; 4; 5 | ||
| + | |style="text-align:right;"|כמו ב' ד' ה‫' | ||
| + | |- | ||
| + | | | ||
| + | *Its property: the product of the greater by the mean is double the product of the greater by the smaller. | ||
| + | :[the general property <math>\scriptstyle a_3\sdot a_2=2\sdot\left(a_3\sdot a_1\right)</math> is incorrect] | ||
| + | |style="text-align:right;"|וסגלת זה שמה שיתקבץ מהכאת הגדול באמצעי כפל המתקבץ מהכאת הגדול בקטן | ||
| + | |- | ||
| + | | | ||
| + | *It follows necessary that the ratio of the mean to the smaller is the double ratio. | ||
| + | :[<math>\scriptstyle a_2:a_1=2</math> is also incorrect] | ||
| + | |style="text-align:right;"|ויתחייב מזה שיהיה לעולם יחס האמצעי אל הקטן הוא יחס הכפל | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ===== The Sixth Proportion ===== | ||
| + | |||
| + | |style="text-align:right;"|ואמנם האמצעי הששי | ||
| + | |- | ||
| + | | | ||
| + | :The second of the two proportions that are opposite to the geometrical proportion. | ||
| + | |style="text-align:right;"|והוא השני לשני המקבילים לאמצעי המדותי | ||
| + | |- | ||
| + | |When there are three terms, the ratio of the excess of the mean over the smaller to the excess of the greater over the mean is as the ratio of the greater to the mean. | ||
| + | :<math>\scriptstyle\left(a_2-a_1\right):\left(a_3-a_2\right)=a_3:a_2</math> | ||
| + | |style="text-align:right;"|הנה הוא כאשר היו שלשה גבולים יחס מותר האמצעי על הקטן אל מותר הגדול אל האמצעי כיחס הגדול אל האמצעי | ||
| + | |- | ||
| + | | | ||
| + | ::Such as: 1; 4; 6 | ||
| + | |style="text-align:right;"|כמו א' ד' ו‫' | ||
| + | |- | ||
| + | | | ||
| + | *Its property: the product of the greater excess by the mean term is equal to the product of the smaller excess by the greater term. | ||
| + | :<math>\scriptstyle\left(a_2-a_1\right)\sdot a_2=\left(a_3-a_2\right)\sdot a_3</math> | ||
| + | |style="text-align:right;"|וסגלת זה שמה שיתקבץ מהכאת המותר הגדול בגבול האמצעי שוה למה שיתקבץ מהכאת המותר הקטן בגבול הגדול | ||
| + | |- | ||
| + | |The proportions that were used from after Pythagoras until Plato's time are the three first proportions. | ||
| + | |style="text-align:right;"|הנה האמצעים הנעשים מאחר פיתאגוריש עד זמן אפלאטון הם השלשה הראשונים | ||
| + | |- | ||
| + | |The other three are their opposites. | ||
| + | |style="text-align:right;"|ואלו השלשה האחרים הם מקבילים | ||
| + | |- | ||
| + | |The four remaining were mentioned by the contemporaries and found to a small extent in the writings of the ancients. | ||
| + | |style="text-align:right;"|ואמנם הארבעה הנשארים אשר זכרום החדשים ומעט מה שימצאו בספרי הקדמונים | ||
| + | |- | ||
| + | |Since they were mentioned literally, it is necessary to mention them - lest the author will be considered ignorant concerning them. | ||
| + | |style="text-align:right;"|וכאשר נזכרו זכר פשוט כבר יחוייב שנזכירם כדי שלא יחשוב חושב שאנו עזבנום להכלותנו בם והם אלו | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ===== The Seventh Proportion ===== | ||
| + | |||
| + | |style="text-align:right;"|האמצעי השביעי | ||
| + | |- | ||
| + | |When there are three terms, the ratio of the excess of the greater over the smaller to the excess of the mean over the smaller is as the ratio of the greater to the smaller. | ||
| + | :<math>\scriptstyle\left(a_3-a_1\right):\left(a_2-a_1\right)=a_3:a_1</math> | ||
| + | |style="text-align:right;"|הוא כאשר היו שלשה גבולים יחס מותר הגדול על הקטן אל מותר האמצעי על הקטן כיחס הגדול אל הקטן | ||
| + | |- | ||
| + | | | ||
| + | ::Such as : 6; 8; 9 | ||
| + | |style="text-align:right;"|כמו ו' ח' ט‫' | ||
| + | |- | ||
| + | | | ||
| + | ===== The Eighth Proportion ===== | ||
| + | |||
| + | |style="text-align:right;"|והאמצעי השמיני | ||
| + | |- | ||
| + | |When the setting of the terms is such that the ratio of the greater to the smaller is as the ratio of the excess of the greater over the smaller to the excess of the greater over the mean. | ||
| + | :<math>\scriptstyle a_3:a_1=\left(a_3-a_1\right):\left(a_3-a_2\right)</math> | ||
| + | |style="text-align:right;"|הנה הוא כאשר היתה הנחת הגבולים כן יחס הגדול אל הקטן כיחס מותר הגדול על הקטן אל מותר הגדול על האמצעי | ||
| + | |- | ||
| + | | | ||
| + | ::Such as: 6; 7; 9 | ||
| + | |style="text-align:right;"|כמו ו' ז' ט‫' | ||
| + | |- | ||
| + | | | ||
| + | ===== The Ninth Proportion ===== | ||
| + | |||
| + | |style="text-align:right;"|והאמצעי התשיעי | ||
| + | |- | ||
| + | |When there are three terms, the ratio of the excess of the greater over the smaller to the excess of the mean over the smaller is as the ratio of the mean to the smaller. | ||
| + | :<math>\scriptstyle\left(a_3-a_1\right):\left(a_2-a_1\right)=a_2:a_1</math> | ||
| + | |style="text-align:right;"|הוא כאשר היו שלשה גבולים יחס מותר הגדול על הקטן אל מותר האמצעי על הקטן כיחס האמצעי אל הקטן | ||
| + | |- | ||
| + | | | ||
| + | ::Such as: 4; 6; 7 | ||
| + | |style="text-align:right;"|כמו ד' ו' ז‫' | ||
| + | |- | ||
| + | | | ||
| + | ===== The Tenth Proportion ===== | ||
| + | |||
| + | |style="text-align:right;"|אמנם האמצעי הי | ||
| + | |- | ||
| + | |When there are three terms, the ratio of the excess of the greater over the smaller to the excess of the greater over the mean is as the ratio of the mean to the smaller. | ||
| + | :<math>\scriptstyle\left(a_3-a_1\right):\left(a_3-a_2\right)=a_2:a_1</math> | ||
| + | |style="text-align:right;"|כאשר היו ג' גבולים יחס מותר הגדול על הקטן אצל מותר הגדול על האמצעי כיחס האמצעי אל הקטן | ||
| + | |- | ||
| + | | | ||
| + | ::Such as: 3; 5; 8 | ||
| + | |style="text-align:right;"|כמו ג' ה' ח‫' | ||
| + | |- | ||
| + | |We have completed all of them in this diagram: | ||
| + | |style="text-align:right;"|והנה השלמנו כלם בזאת הצורה | ||
| + | |- | ||
| + | |} | ||
| + | |||
| + | |||
| + | {|class="wikitable" style="margin-left: auto; margin-right: auto; color:blue" | ||
| + | |- | ||
| + | |its property: equality of the difference and equality of the sum of the two extremes to double the mean | ||
| + | |3 | ||
| + | |2 | ||
| + | |1 | ||
| + | |arithmetic proportion | ||
| + | |- | ||
| + | |its property: equality of the ratio and the ratio of the mean to the smaller is as the ratio of the difference between the two greater to the difference between the two smaller | ||
| + | |4 | ||
| + | |2 | ||
| + | |1 | ||
| + | |geometric proportion | ||
| + | |- | ||
| + | |its property: the ratio of the greater to the smaller is as the ratio of the difference between the two greater to the difference between the two smaller | ||
| + | |6 | ||
| + | |4 | ||
| + | |3 | ||
| + | |harmonic proportion | ||
| + | |- | ||
| + | |its property: the ratio of the greater to the smaller is as the ratio of the difference between the two smaller to the difference between the two greater | ||
| + | |6 | ||
| + | |5 | ||
| + | |3 | ||
| + | |opposite to the harmonic proportion | ||
| + | |- | ||
| + | |its property: the ratio of the mean to the smaller is as the ratio of the difference between the two smaller to the difference between the two greater | ||
| + | |5 | ||
| + | |4 | ||
| + | |2 | ||
| + | |opposite to the geometric proportion | ||
| + | |- | ||
| + | |its property: the ratio of the greater to the mean is as the ratio of the difference between the two smaller to the difference between the two greater | ||
| + | |6 | ||
| + | |4 | ||
| + | |1 | ||
| + | |opposite to the geometric proportion | ||
| + | |- | ||
| + | |its property: the ratio of the excess of the greater over the smaller to the excess of the mean over the smaller is as the ratio of the greater to the smaller | ||
| + | |9 | ||
| + | |8 | ||
| + | |6 | ||
| + | |seventh proportion | ||
| + | |- | ||
| + | |its property: the ratio of the greater to the smaller is as the ratio of the excess of the greater over the smaller to the excess of the greater over the mean | ||
| + | |9 | ||
| + | |7 | ||
| + | |6 | ||
| + | |eighth proportion | ||
| + | |- | ||
| + | |its property: the ratio of the excess of the greater over the smaller to the excess of the mean over the smaller is as the ratio of the mean to the smaller | ||
| + | |7 | ||
| + | |6 | ||
| + | |4 | ||
| + | |ninth proportion | ||
| + | |- | ||
| + | |its property: the ratio of the excess of the greater over the smaller to the excess of the greater over the mean is as the ratio of the mean to the smaller | ||
| + | |8 | ||
| + | |5 | ||
| + | |3 | ||
| + | |tenth proportion | ||
| + | |} | ||
| + | |||
| + | {|class="wikitable" style="margin-left: auto; margin-right: auto; text-align:center;" | ||
| + | |- | ||
| + | |style="text-align:right;"|סגולתו שווי היתרון ושווי שני הקצוות מקובצים לאמצעי כפול | ||
| + | |style="text-align:right;"|ג | ||
| + | |style="text-align:right;"|ב | ||
| + | |style="text-align:right;"|א | ||
| + | |style="text-align:right;"|{{#annot:term|1769,1743|qHu7}}האמצעי החשבוני{{#annotend:qHu7}} | ||
| + | |- | ||
| + | |style="text-align:right;"|סגולתו שווי היחס ושיחס האמצעי לקטן כיחס מותר מה שבין שני הגדולים אל המותר שבין הקטנים | ||
| + | |style="text-align:right;"|ד | ||
| + | |style="text-align:right;"|ב | ||
| + | |style="text-align:right;"|א | ||
| + | |style="text-align:right;"|האמצעי המדותי | ||
| + | |- | ||
| + | |style="text-align:right;"|סגולתו שיחס הגדול אל הקטן כיחס מותר מה שבין שני הגדולים אל המותר שבין שני הקטנים | ||
| + | |style="text-align:right;"|ו | ||
| + | |style="text-align:right;"|ד | ||
| + | |style="text-align:right;"|ג | ||
| + | |style="text-align:right;"|האמצעי החבוריי | ||
| + | |- | ||
| + | |style="text-align:right;"|סגולתו שיחס הגדול לקטן כיחס מותר מה שבין שני הקטנים אל המותר שבין שני הגדולים | ||
| + | |style="text-align:right;"|ו | ||
| + | |style="text-align:right;"|ה | ||
| + | |style="text-align:right;"|ג | ||
| + | |style="text-align:right;"|המקביל לחבוריי | ||
| + | |- | ||
| + | |style="text-align:right;"|סגולתו שיחס האמצעי אל הקטן כיחס מותר מה שבין שני הקטנים אל מותר שבין שני הגדולים | ||
| + | |style="text-align:right;"|ה | ||
| + | |style="text-align:right;"|ד | ||
| + | |style="text-align:right;"|ב | ||
| + | |style="text-align:right;"|המקביל למדותיי | ||
| + | |- | ||
| + | |style="text-align:right;"|סגולתו שיחס הגדול לאמצעי כיחס המותר שבין שני הקטנים אל מותר שבין שני הגדולים | ||
| + | |style="text-align:right;"|ו | ||
| + | |style="text-align:right;"|ד | ||
| + | |style="text-align:right;"|א | ||
| + | |style="text-align:right;"|המקביל ג"כ למדותיי | ||
| + | |- | ||
| + | |style="text-align:right;"|סגולתו שיחס מותר הגדול על הקטן אל מותר האמצעי על הקטן כיחס הגדול אל הקטן | ||
| + | |style="text-align:right;"|ט | ||
| + | |style="text-align:right;"|ח | ||
| + | |style="text-align:right;"|ו | ||
| + | |style="text-align:right;"|האמצעי השביעי | ||
| + | |- | ||
| + | |style="text-align:right;"|סגולתו שיחס הגדול לקטן כיחס מותר הגדול על הקטן אל מותר הגדול על האמצעי | ||
| + | |style="text-align:right;"|ט | ||
| + | |style="text-align:right;"|ז | ||
| + | |style="text-align:right;"|ו | ||
| + | |style="text-align:right;"|האמצעי השמיני | ||
| + | |- | ||
| + | |style="text-align:right;"|סגולתו שיחס מותר הגדול על הקטן אל מותר האמצעי על הקטן כיחס האמצעי אל הקטן | ||
| + | |style="text-align:right;"|ז | ||
| + | |style="text-align:right;"|ו | ||
| + | |style="text-align:right;"|ד | ||
| + | |style="text-align:right;"|האמצעי התשיעי | ||
| + | |- | ||
| + | |style="text-align:right;"|סגולתו שיחס מותר הגדול על הקטן אל מותר הגדול על האמצעי כיחס האמצעי אל הקטן | ||
| + | |style="text-align:right;"|ח | ||
| + | |style="text-align:right;"|ה | ||
| + | |style="text-align:right;"|ג | ||
| + | |style="text-align:right;"|האמצעי העשירי | ||
| + | |} | ||
| + | |||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== The Perfect Proportion that Encompasses the body ==== | ||
| + | |||
| + | !style="text-align:right;"|הדבור באמצעי השלם המקיף במוגשם | ||
| + | |- | ||
| + | |The proportion that has three terms, as the span of its ends is composed of two intervals, which are: | ||
| + | |style="text-align:right;"|האמצעי בעל הג' גבולים מפני שהקפת תכליותיו מורכבת מב' המרחקים והם | ||
| + | |- | ||
| + | | | ||
| + | *the interval of what is between the greater and the mean. | ||
| + | |style="text-align:right;"|מרחק מה שבין הגדול והאמצעי | ||
| + | |- | ||
| + | | | ||
| + | *the interval of what is between the mean and the smaller | ||
| + | |style="text-align:right;"|ומרחק מה שבין אמצעי והקטן | ||
| + | |- | ||
| + | |As in the surface that is composed of length and breadth. | ||
| + | |style="text-align:right;"|כמו אותם אל הפשוט אשר הוא מורכב מהארך והרחב | ||
| + | |- | ||
| + | |The proportions that are imagined in the solid body are more perfect proportions, and it is impossible that there would be a proportion that deserves to be named perfect more than them, as the perfection of the solid body is complete, since it is impossible by nature that it will get more than three dimensions, which are the length, the breadth, and the depth. | ||
| + | |style="text-align:right;"|ואמנם האמצעים המדומים בגשם הנה הם יותר שלמים האמצעיים וא"א להיות אמצעי יותר ראוי בשם השלימות מהם כמו שהמוגשם כבר יכלה שלמותו אחר שאי אפשר בטבע שיקבל תוספת על המרחקים הג' אשר הם הארך והרחב והעומק | ||
| + | |- | ||
| + | |Therefore, the proportion that has four terms, as the span of its ends is composed of three intervals, which are: | ||
| + | |style="text-align:right;"|הנה אם כן האמצעי בעל הד' גבולים מפני שההקפה אשר לתכליותיו היא מורכבת מג' מרחקים והם | ||
| + | |- | ||
| + | | | ||
| + | *the interval between the first and the second. | ||
| + | |style="text-align:right;"|מרחק מה שבין הראשון והב‫' | ||
| + | |- | ||
| + | | | ||
| + | *the interval between the second and the third. | ||
| + | |style="text-align:right;"|ומרחק מה שבין הב' לשלישי | ||
| + | |- | ||
| + | | | ||
| + | *the interval between the third and the fourth. | ||
| + | |style="text-align:right;"|ומרחק מה שבין הג' לד‫' | ||
| + | |- | ||
| + | |It is by reason and necessary the perfect proportion imagined in the body. | ||
| + | |style="text-align:right;"|היה בדין ובראוי הוא האמצעי השלם הדומה במוגשם | ||
| + | |- | ||
| + | |It has this analogy by reason, since the three dimensions of the body are in four limits, which are the unit, the side, the surface, and the body. | ||
| + | |style="text-align:right;"|ובדין לו זה הדמיון כי היו מרחקי הגשם הג' אמנם עמדו מד' גבולים והם האחדות והצלע והפשוט והגשם | ||
| + | |- | ||
| + | |For the space between the unit and the body is the body itself and it consists of the three intervals, which are: the intervals between the unit and the side, the intervals between the side and the surface, and the intervals between the surface and the body. | ||
| + | |style="text-align:right;"|כי מרחק מה שבין האחדות והגשם הוא המוגשם עצמו והוא מורכב מהג' רחקים אשר הם מרחק מה שבין האחדות והצלע ומרחק מה שבין הצלע והפשוט ומרחק מה שבין הפשוט והגשם | ||
| + | |- | ||
| + | |Also the perfect proportion that has four limits is the span of its ends, and one finds that this span consists of the three intervals, which are: the interval from the first term to the second, the interval from the second to the third, and the interval from the third to the fourth. | ||
| + | |style="text-align:right;"|וכן האמצעי השלם בעל הד' גבולים הוא הקפת תכליותיו ותמצא אותה ההקפה מורכבת מג' המרחקים אשר הם מרחק הגבול הראשון מהב' ומרחק הב' מהג' והמרחק הג' מהד‫' | ||
| + | |- | ||
| + | |Nicomachus brought an example for this perfect solid comprehensive proportion after demonstrating that the comprehension is by the properties of the three proportions, i.e. the arithmetic proportion, the geometric proportion, and the harmonic proportion. | ||
| + | |style="text-align:right;"|וכבר הניח ניקומכוש לזה האמצעי השלם המוגשם המקיף דמיון אחר הראנו כי ההקפה בסגולות האמצעיים הג' ר"ל אמצע החשבון ואמצע המדות אמצע החבור | ||
| + | |- | ||
| + | |This is the diagram of the example that Nicomachus brought: | ||
| + | |style="text-align:right;"|וזה צורתו המשל שהביא ניקומאכוש | ||
| + | |- | ||
| + | | | ||
| + | ::{|class="wikitable" style="color: blue; text-align:center;" | ||
| + | |- | ||
| + | |12||9||8||6 | ||
| + | |} | ||
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | |יב||ט||ח||ו | ||
| + | |} | ||
| + | |||
| + | |- | ||
| + | | | ||
| + | *The property of the arithmetic proportion in this perfect solid comprehensive proportion is seen in three of the terms, which are the first, the third, and the fourth [= 6; 9; 12], since the excess of the fourth over the third is the excess of the third over the first; also the sum of the first and the fourth is as double the mean, and this is the mentioned property. Since this is so, all the properties mentioned above for the arithmetic proportion are affirmed in it. | ||
| + | :[<math>\scriptstyle{\color{blue}{a_4-a_3=12-9=9-6=a_3-a_1}}</math>] | ||
| + | :[<math>\scriptstyle{\color{blue}{a_1+a_4=6+12=2\sdot9=2\sdot a_3}}</math>] | ||
| + | |style="text-align:right;"|ואמנם סגולת האמצעי החשבון הנמצאת בזה האמצעי השלם המוגשם המקיף הנה יראה בג' גבולי' מהם והם הראשון והג' והד' וזה לפי שמותר הרביעי על הג' הוא מותר הג' על הראשון ושהראשון והד' מקובצים כמוכפל האמצעי והיא הסגולה שזכרנו וכאשר היה זה כן הנה כבר יתחייבו לו כל הסגולות אשר קדם זכרם לאמצע החשבון | ||
| + | |- | ||
| + | | | ||
| + | *The property of the geometric proportion in this perfect solid comprehensive proportion is seen in the four terms together [= 6; 8; 9; 12], when the first is related to the third, and the second to the fourth. This is because the ratio will then be one, and the product of the two extremes one by the other is as the product of the two means one by the other. Since this is so, the rest of the properties of the geometric proportion are affirmed it. | ||
| + | :[<math>\scriptstyle{\color{blue}{a_1:a_3=6:9=8:12=a_2:a_4}}</math>] | ||
| + | :[<math>\scriptstyle{\color{blue}{a_1\sdot a_4=6\sdot12=8\sdot9=a_2\sdot a_3}}</math>] | ||
| + | |style="text-align:right;"|ואמנם סגולת אמצע המדות הנמצאת בזה האמצעי השלם המוגשם המקיף תראה בגבולי' הד' יחד כאשר יוחס הראשון אל הג' והב' אל הד' וזה לפי שהיחס יהיה אז אחד ויהיה מוכה ב' הקצוות א' מהם באחר כמוכה ב' האמצעיי' א' מהם באחר וכאשר היה זה כן יתחייבו לו שאר הסגולות הנמצאות לאמצעי המדות | ||
| + | |- | ||
| + | | | ||
| + | *The property of the harmonic proportion in this perfect solid comprehensive proportion is seen in three of these terms, as seen in the arithmetic proportion, and the evidence of this is when the fourth is related to the second, and the second to the first [= 6; 8; 12], for then the ratio of the excess of the fourth over the second to the fourth is as the ratio of the excess of the second over the first [to the first]. Since this is so, the rest of the properties in the harmonic proportion are also affirmed in it. | ||
| + | :[<math>\scriptstyle{\color{blue}{\left(a_4-a_2\right):a_4=\left(12-8\right):12=\left(8-6\right):6=\left(a_2-a_1\right):a_1}}</math>] | ||
| + | |style="text-align:right;"|ואמנם סגולת האמצעי החבור אשר תמצא בזה האמצעי השלם המוגשם המקיף תראה בג' מגבוליו כמו שנראה באמצעי החשבון והראות זה יהיה כאשר ייוחס הד' אל הב' והב' אל הראשון כי יהיה אז יחס מותר הד' על הב' אל הד' כיחס מותר הב' על הראשון וכאשר היה זה כן הנה יתחייבו לו ג"כ שאר הסגולות הנמצאו' אל אמצעי החבור | ||
| + | |- | ||
| + | |The properties of the arithmetic proportion are evident in three terms, likewise the properties of the harmonic proportion are in three terms, however the evidence of the properties of the geometric proportion is different, as they are evident when four terms are applied together, since the virtue of these is mean between the two other techniques and it takes the appropriate from both together, thus what is different in them is summed by it. | ||
| + | |style="text-align:right;"|ואמנם נראו סגולות אמצעי החשבון בג' גבולים וסגולות אמצעי החבור בג' גבולים וחלוף זה הראות סגולות אמצעי המדות כאשר השתמש בהראותם ד' גבולים יחד לפי שהמדה באלו היא אמצעית בין ב' המלאכות האחרות ותקח הראוי מהן יחד והנה יתקבץ בה מה שתפריש בם | ||
| + | |- | ||
| + | |Apart than that, we say that all the harmonies that exist in the art of music are evident in this perfect solid comprehensive proportion: | ||
| + | |style="text-align:right;"|וזולת זה אנו נאמר כי כבר יראה בזה האמצעי השלם המוגשם המקיף כל ההסכמות הנמצאות במלאכת החבור | ||
| + | |- | ||
| + | | | ||
| + | *The diatessaron, which is in the sesquitertian ratio, is seen in the ratio of the first to the second, as well as the ratio of the third to the fourth. | ||
| + | :[<math>\scriptstyle{\color{blue}{a_2:a_1=8:6=1+\frac{1}{3}=12:9=a_4:a_3}}</math>] | ||
| + | |style="text-align:right;"|אם ההסכמה בד' והיא על {{#annot:sesquitertian|125|ctVx}}יחס דמיון ושליש{{#annotend:ctVx}} תראה מהקשת הראשון אל הב' ותרא' ג"כ מהקשת הג' אל הד‫' | ||
| + | |- | ||
| + | | | ||
| + | *The diapente, which is in the sesquialter ratio, is seen in the ratio of the first to the third, as well as the ratio of the second to the fourth.. | ||
| + | :[<math>\scriptstyle{\color{blue}{a_3:a_1=9:6=1+\frac{1}{2}=12:8=a_4:a_2}}</math>] | ||
| + | |style="text-align:right;"|ואמנם ההסכמה בחומש והוא על {{#annot:sesquialter|125|NHoQ}}יחס דמיון וחצי{{#annotend:NHoQ}} תרא' מהקשת הראשון אל הג' ותראה גם כן מהקשת השני אל הרביעי | ||
| + | |- | ||
| + | | | ||
| + | *The diapason, which is in the double ratio, is seen in the ratio of the first to the fourth. | ||
| + | :[<math>\scriptstyle{\color{blue}{a_4:a_1=12:6=2}}</math>] | ||
| + | |style="text-align:right;"|ואמנם ההסכמה בכל והוא על {{#annot:double ratio|124|VNE8}}יחס הכפל השניי{{#annotend:VNE8}} תראה מהקשת הראשון אל הד‫' | ||
| + | |- | ||
| + | | | ||
| + | *The interval of a tone, which is in the sesquioctavian ratio, is seen in the ratio of the second and the third. | ||
| + | :[<math>\scriptstyle{\color{blue}{a_3:a_2=9:8=1+\frac{1}{8}}}</math>] | ||
| + | |style="text-align:right;"|ואמנם הזמן והוא על {{#annot:sesquioctavian|125|sVbr}}יחס דמיון ושמינית{{#annotend:sVbr}} תראה מהקשת מה שבין הב' והג‫' | ||
| + | |- | ||
| + | | | ||
| + | :This interval of a tone is a measure common to all melodies, and it is the smallest interval in the melodies. | ||
| + | |style="text-align:right;"|וזה הזמן הוא שעור משותף לכל הליחנים והוא הפחות שבמרחקים המקבילים בליחנים להחלק | ||
| + | |- | ||
| + | | | ||
| + | :It is the difference between the diapente and the diatessaron, as the superoctave is the difference between the sesquialter and the sesquitertian | ||
| + | :[<math>\scriptstyle{\color{blue}{\left(1+\frac{1}{2}\right):\left(1+\frac{1}{3}\right)=1+\frac{1}{8}}}</math>] | ||
| + | |style="text-align:right;"|והוא היתרון אשר בין ההסכמה בחומש וההסכמה בד' כמו שהשמינית הוא המותר בין המוסיף חצי ובין המוסיף שליש | ||
| + | |- | ||
| + | |I have concluded showing these harmonies in this perfect solid comprehensive proportion in this diagram. | ||
| + | |style="text-align:right;"|וכבר השלמתי לך איך יראו אלו ההסכמות מזה האמצעי השלם המקיף המוגשם בצורה זו | ||
| + | |- | ||
| + | |Over and done. | ||
| + | |style="text-align:right;"|תם ונשלם | ||
| + | |- | ||
| + | |Praise to God, the Creator of the world. | ||
| + | |style="text-align:right;"|שבח לאל בורא עולם | ||
| + | |||
| + | |- | ||
| + | | | ||
| + | |} | ||
| + | |||
| + | == Appendix: Bibliography == | ||
| + | '''Nicomachus of Gerasa'''<br> | ||
| + | 2nd century C. E<br> | ||
| + | '''''’Αριθμητικής είσαγωγής βιβλία β – Introduction to Arithmetic'''''<br> | ||
| + | |||
| + | |||
| + | '''<u>Critical Edition</u>''': | ||
| + | *Nicomachus of Gerasa. Introduction to Arithmetic. trans. Martin L. D'Ooge. Chicago IL: Encyclopaedia Britannica, 1955.[Great Books of the Western World, vol.11], pp. 811 – 848.<br> | ||
| + | |||
| + | |||
| + | – Hebrew translation –<br> | ||
| + | '''Qalonymos ben Qalonymos''' (known as Maestro Calo or Callus)<br> | ||
| + | South of France, 1286/7-after 1329<br> | ||
| + | '''''Sefer ha-Aritmitiqa / Aritmaiti'''''<br> | ||
| + | 1317<br> | ||
| + | |||
| + | |||
| + | |||
| + | <u>'''Manuscripts</u>:'''<br> | ||
| + | :1) Halle, Universitätsbibliothek Yb Qu. 5/1 (IMHM: f 71790), ff. 1r-54r (15th century) | ||
| + | :2) München, Bayerische Staatsbibliothek, Cod. hebr. 36/14 (IMHM: f 1166), ff. 144r-164r (Istanbul, 1485) | ||
| + | ::[[https://daten.digitale-sammlungen.de/0009/bsb00095507/images/index.html?id=00095507&groesser=&fip=eayayztsxdsydeayaeayaxseayaenxdsydxdsydfsdr&no=10&seite=306 München36]] | ||
| + | :3) New York, Jewish Theological Seminary Ms. 2449/2 (IMHM: f 28702), ff. 107r-153r (15th century) | ||
| + | ::[[https://digitalcollections.jtsa.edu/islandora/object/jts:257709#page/217/mode/2up JTS2449]] | ||
| + | :4) Paris, Bibliothèque Mazarine 4478/1 (IMHM: f 4414), ff. 194-296 (15th century) | ||
| + | :5) Paris, Bibliothèque Nationale de France heb. 1028/1 (IMHM: f 15720), ff. 1r-54r (1342) | ||
| + | ::[[https://gallica.bnf.fr/ark:/12148/btv1b90646735/f3.image Paris1028]] | ||
| + | :6) Paris, Bibliothèque Nationale de France heb. 1029/1 (IMHM: f 15721), ff. 1r-30v (15th – 16th century) | ||
| + | ::[[https://gallica.bnf.fr/ark:/12148/btv1b9064672r/f2.image Paris1029]] | ||
| + | :7) Paris, Bibliothèque Nationale de France heb. 1093/2 (IMHM: f 15043), ff. 127r-155v (15th century) | ||
| + | ::[[https://gallica.bnf.fr/ark:/12148/btv1b10550866s/f266.image Paris1093]] | ||
| + | :8) Paris, Bibliothèque Nationale de France heb. 1095/8 (IMHM: f 15045), ff. 177-226 (15th century) | ||
| + | ::[[https://gallica.bnf.fr/ark:/12148/btv1b10545036x/f370.image Paris1095]] | ||
| + | <br> | ||
| + | |||
| + | <span style=color:blue>The transcript is based mainly on manuscript Halle</span> | ||
| + | |||
| + | '''<u>Bibliography</u>:'''<br> | ||
| + | *Freudenthal, Gad et Lévy Tony. 2004. De Gérase à Bagdad: Ibn Bahriz, al-Kindi, et leur recension arabe de l’Introduction arithmétique de Nicomaque, d’après la version hébraïque de Qalonymos ben Qalonymos d’Arles, In R. Morelon et A. Hasnawi eds. De Zénon d’Elée à Poincaré. Recueil d’études en hommage à Roshdi Rashed. Louvain-Paris. pp. 479-544. | ||
| + | *Freudenthal, Gad and Mauro Zonta. 2007. Remnants of Habīb ibn Bahrīz’s Arabic translation of Nicomachus of Gerasa’s Introdaction to Arithmetic, in Y. Tzvi Langermann and Josef Stern eds. Adaptations and Innovations: Studies on the interaction between Jewish and Islamic thought and literature from the early Middle Ages to the late twentieth century, dedicated to Professor Joel L. Kraemer. Paris, Louvain and Dudley: Peeters, 2007. pp.67-82. | ||
| + | *———. 2009. Nicomachus of Gerasa in Spain, Circa 1100: Abraham bar Ḥiyya’s Testimony, Aleph 9.2 (2009), pp. 189-224. | ||
| + | *Langermann, Y. Tzvi. 2001. Studies in Medieval Hebrew Pythagoreanism: Translations and Notes to Nicomachus; Arithmological Texts, Micrologus IX, pp. 219–36. | ||
| + | *Sarfatti, Gad ben ‛Ami. 1968. Mathematical Terminology in Hebrew Scientific Literature of the Middle Ages. Jerusalem: Magnes Press, pp. 200-214. | ||
| + | *Steinschneider, Moritz. 1894. Miscellen 26. Nikomachus, Arithmetik, Monatsschrift für die Geschichte und Wissenschaft des Judenthums 38, pp. 68–77.<br> | ||
| + | |||
| + | |||
| + | – '''Summary''' – <br> | ||
| + | <u>'''Manuscript</u>:'''<br> | ||
| + | *Oxford, Bodleian Library MS Mich. 29/3 (IMHM: f 20994), ff. 131v-158r (cat. Neub. 2302, 3; 18th century)<br> | ||
| + | |||
| + | |||
| + | – '''Commentaries on the Introduction to Arithmetic by Nicomachus''' – <br> | ||
| + | |||
| + | '''<u>Caleb Afendopolo</u>'''<br> | ||
| + | Constantinople, 1499<br> | ||
| + | |||
| + | |||
| + | <u>'''Manuscript</u>:'''<br> | ||
| + | *Berlin, Staatsbibliothek (Preußischer Kulturbesitz) Or. Qu. 760 (IMHM: f 1742) (1499) | ||
| + | :[[https://digital.staatsbibliothek-berlin.de/werkansicht?PPN=PPN767433874&PHYSID=PHYS_0017&DMDID= Berlin760]] | ||
| + | |||
| + | '''<u>Bibliography</u>:'''<br> | ||
| + | *Steinschneider, Moritz. 1896. Miscellen 36. Kaleb Afednopolo's encyklopädische Einteilung der Wissenschaften, Monatsschrift für die Geschichte und Wissenschaft des Judenthums 40, pp. 90–94.<br> | ||
| + | |||
| + | |||
| + | |||
| + | '''<u>A certain Yosef</u>'''<br> | ||
| + | |||
| + | <u>'''Manuscript</u>:'''<br> | ||
| + | *Moscow, Russian State Library, Ms. Guenzburg 340/1 (IMHM: f 47750), ff. 2r-18v (16th century) | ||
| + | :[[https://web.nli.org.il/sites/NLI/Hebrew/digitallibrary/pages/viewer.aspx?&presentorid=MANUSCRIPTS&docid=PNX_MANUSCRIPTS000069389-1# Guenzburg340]] | ||
| + | |||
| + | |||
| + | '''<u>Abraham Yerushalmi</u>'''<br> | ||
| + | |||
| + | <u>'''Manuscripts</u>:'''<br> | ||
| + | *Moscow, Russian State Library, Ms. Guenzburg 340/2 (IMHM: f 47750), ff. 19r-23r (16th century) | ||
| + | :[[https://web.nli.org.il/sites/NLI/Hebrew/digitallibrary/pages/viewer.aspx?&presentorid=MANUSCRIPTS&docid=PNX_MANUSCRIPTS000069389-1# Guenzburg340]] | ||
Latest revision as of 21:12, 17 December 2022
Contents
- 1 Book One
- 1.1 [Prologue by al-Kindī's Student]
- 1.2 Introduction
- 1.3 [Absolute Quantity - Arithmetic]
- 1.3.1 The Discussion on the Nature of Number
- 1.3.2 The Discussion on the Definition of Number and its Categorization
- 1.4 The Discussion of the Quality of the Relative Quantity and its Division into the Equal and the Unequal
- 2 Book Two
- 2.1 The First Section: Reducing the Ratios to Equality
- 2.2 The Discussion on the Arrangement of the Terms of each Ratio of two Given Numbers from Superparticulars
- 2.3 [Absolute Quantity]
- 2.3.1 The Discussion of the Quality of the Absolute Numbers that are Potentially Commensurable with the Geometric Shapes
- 2.3.1.1 Plane Numbers
- 2.3.1.1.1 The Discussion of the Aspect of Similarity between the Number to the Line
- 2.3.1.1.2 The Discussion of the Triangle as the Foundation of all Rectilinear Surfaces
- 2.3.1.1.3 The Discussion of the Triangular Numbers, their Production and their Sides
- 2.3.1.1.4 The Discussion of the Square Numbers, their Sides and their Production
- 2.3.1.1.5 The Discussion on the Pentagonal Numbers, their Production and their Sides
- 2.3.1.1.6 The Discussion about how All Plane Polygonals Consist of and are Resolved into Triangles
- 2.3.1.2 The Discussion on the Solid Numbers
- 2.3.1.2.1 The Discussion on the Cube Number
- 2.3.1.2.2 The Discussion on the Scalene Numbers
- 2.3.1.2.3 The Discussion on the Parallelepipedon Numbers
- 2.3.1.2.4 The Discussion on the Natural Root of the Numerical “Sameness” and Numerical “Otherness”
- 2.3.1.2.5 The Discussion on the Brick Numbers
- 2.3.1.2.6 The Discussion on the Circular and Spherical Numbers
- 2.3.1.1 Plane Numbers
- 2.3.2 The Discussion of the Indications of the Ancients that the Beginning of Numbers is similar to the Beginning of the World
- 2.3.3 Properties of the species of Numbers and their Mutual Harmony
- 2.3.1 The Discussion of the Quality of the Absolute Numbers that are Potentially Commensurable with the Geometric Shapes
- 2.4 [Relative Quantity]
- 2.4.1 The Discussion on The Ten Means
- 2.4.1.1 The Arithmetic Proportion
- 2.4.1.2 The Geometic Proportion
- 2.4.1.3 The Harmonic Proportion
- 2.4.1.4 [Extracting a Third Term in the Arithmetic, Geometric, and Harmonic Proportions]
- 2.4.1.5 The Fourth Proportion
- 2.4.1.6 The Fifth Proportion
- 2.4.1.7 The Sixth Proportion
- 2.4.1.8 The Seventh Proportion
- 2.4.1.9 The Eighth Proportion
- 2.4.1.10 The Ninth Proportion
- 2.4.1.11 The Tenth Proportion
- 2.4.2 The Perfect Proportion that Encompasses the body
- 2.4.1 The Discussion on The Ten Means
- 3 Appendix: Bibliography
Book One |
המאמר הראשון מספר הארתמאיטי |
|---|---|
[Prologue by al-Kindī's Student] |
|
| May God fulfill your wishes, carry out your wishes and increase His grace and His pleasantness upon you. | ימלא הבורא משאלותיך ויפיק זממיך ויוסיף חסדו ונעמו אצלך |
| [The history of the successive translations of the text:] | |
| I have understood - may God perpetuate your glory - what you have mentioned: that you had studied the famous arithmetic book that is among us, | הבינותי יתמיד האל כבודך מה שזכרת כי עיינת בספר הארתמאיטיקא הידוע אשר בינינו |
|
אשר הניחו וחברו ניקומאכוש אלגהר שיני הפיתאגורי |
|
בנסחא אשר תקננו זה הספר ממנה על דעת מלמדנו המעולה יעקב בן אסחק אלצבאה אלכנדי |
|
משבש מחשבות חביב בן בהריז אלנסטורי אשר תרגמו מהסריאני אל הערבי לבעל שני הימינים טאהר בן אלחסין |
| You have wished to revise it in the first section, from the place of the numbers and onwards, for it was indeed revised from this place, since it has already been preceded by that which in the science of logic could help him to understand the previous introduction, before the mentioning of the number, and this introduction did not reach you and you did not know its purpose nor what its author has mentioned in it. | ואתה ראית לתקנו במאמר הראשון ממקום המספרים והלאה לפי שהוא אמנם תוקן מזה המקום לפי שכבר קדם לו בחכמת ההגיון מה שיעזרהו להבין פתיחתו הקודמת לפני זכירת המספר ושזאת הפתיחה לא נפלה אליך ולא ידעת כוונתה ולא מה זכר בה מניחה |
| What you have asked for in this respect, is explained to you, as I explained it in our other explanations on the book. | ואשר שאלת מזה הנו מפורש לך כמו שפירשתי בשאר פירושינו הנשארים בספר |
| We have summarized his remarks in a concise discussion, without lengthiness or repetitions. | וקצרינו לעניניו במאמר קצר מבלתי אריכות והכפלה |
| You think it a precious benefit and a wonderful guidance. | ואתה חושב שבזה תועלת יקרה והערה נפלאה |
| But I inform you, may God grant you honor, that many times I perhaps wished to describe something in detail and at length, in order to reach the intention of the author of the book. | ואני מודיעך יכבדך האל אולי ארצה הרבה פעמים לתאר דבר בהפלגה ואריכות כדי להגיע בו אל כוונת מניח הספר |
| Then, I came across a concise statement I heard from our master, Abū Yusūf, who already succeeded in explaining what you had requested to explain and in interpreting what you had intended to interpret, in spite of his brevity, as a long speech would not have achieved and a detailed description would not have surpassed. | ויזדמן לי מאמר קצר שמעתיו ממלמדנו אבו יוסף לבאר מה ששאלת לבארו ולפרש מה שכוונת לפרשו כבר הגיע ממנו עם קצורו כאשר לא יגיעהו מאמר מאריך ולא יעברהו תאר מפליג |
| So, I have abandoned what I wanted to reveal through long words, in favor of his concise speech, and because of this, I will refrain from embellishing the book and decorating it more. | ואעזוב מה שראיתי לגלותו בדברים מרובים בעבור מאמרו הקצר ובעבור זה אמנע אני מליפות הספר ולקשטו ביותר |
| More than once I have heard our master saying that the philosophy of these two men, i.e. Ptolemy and Nicomachus, is best clarified in the introduction to their works - Ptolemy in his introduction to the Almagest and Nicomachus in the introduction to the arithmetic book - for the introductions of these two works stimulate elevated regions of philosophy and they are of valuable virtue in knowledge. | וממה ששמעתיו ממלמדנו יותר מפעם אחת כשיאמר שהיותר ראוי ממה שתתבאר בו פילוסופית שני אלו האנשים ר"ל בטלמיוס וניקומאכוש הוא בפתיחת נושאיהם אם בטלמיוס בפתיחתו לספר המגסטי ואם ניקומאכוש בפתיחת ספר הארתמאיטיקא כי פתיחות שני אלו הספרים מעוררות על מקומות עליונים בפילוסופייא ומדרגתם יקרה בידיעה |
| Your thought concerning this was not erroneous. Indeed, I thought, by God, that it is true, because the considerable scope of the introduction of this book and the virtue of the quantity of its benefit and goodness are truly comprehended by you. | ולא כזבה מחשבתך בזה ואמנם אחשוב אותה חי השם שהיא אמתית למה שהתאמת אצלך גודל מעלת פתיחת זה הספר ומעלת שעור תועלתו וטובו |
| In answer to your request, may God glorify you, drawn to appease your mind with anything I can avail for you. | ואני השיבותיך יפארך השם למה ששאלת נמשך להפיס דעתך בכל מה שאוכל להועילך |
| I wrote to you the introduction of this book in my own work, here, with all that can be explained of it, completing the sufficient speech and omitting any repetition and redundancy. | וכתבתי אליך פתיחת זה הספר בספרי זה וכל מה שאפשר לבארו ממנו בהשלמת המאמר המספיק ולהשליך ההכפלה והמותר |
| I explained its terms and removed from it its obscurity and difficulty. | ואפרש בו מלותיו והסירותי ממנו עמקו וקשיו |
| In doing so, I made it more accessible to you than the words of the translator, so that there will be no difference between the meanings. | וכבר עשיתי זה והקרבתיו לך ממלת המעתיק אותו מבלתי שיהיה בין הענינים חלוף |
| Indeed, what is of a high virtue is far from the custom. | ואמנם מה שהיה עליון במדרגה רחוק מהמנהג |
| I will not depart from the order of the author of this book. | הנני לא אסור מלספר סדר מניח הספר |
| But I append to each chapter in this description a discussion attached to that chapter that states the true meaning of the thesis. | אבל אני ממשיך לכל פרק שיהיה בזה התאר מאמר סמוך לאותו הפרק מגיד אמתת הדרוש |
| I shall not pass beyond the opinion of our master, Abū Yusūf, concerning any thing, to which I will refer. | ולא אעבור בדבר ממה שאביא בו דעת מלמדנו אבו יוסף |
| Now it is the time to begin with what you asked for. | ובכאן עת להתחיל במה ששאלת |
| May you succeed in that. | והיה בו מצליח |
| May God, the exalted, lead you to what will save your soul. | יישירך השם יתע' למה שתושע בו נפשך |
Introduction |
|
| Nicomachus said: The first among the ancients who scrutinized the science and deepened in it - the first of them was Pythagoras - defined philosophy by saying that philosophy is the the love of science, as its name indicates this, for its translation is "the love of science". | אמר ניקומאכוש שהקדמונים הראשונים אשר דקדקו בחכמה והעמיקו בה והיה הראשון להם פיתאגורש גדרו הפילוסופייא כשאמרו שהפילוסופיאה אהבת החכמה כמו ששמה מורה על זה ממנה כי העתקתו אהבת החכמה |
| By saying "as its name indicates this", he meant that the term philosophy is composed of two words "filo" and "sofia" - "filo" is loving and "sofia" is science - so the term is composed from "loving science". | ירצה באומרו שמה מורה על זה ממנה לפי ששם הפילוסופיאה מורכב משתי מלות פילו וסופיה ופילו אוהב וסופיה חכמה הנה שמה מורכב מאוהב החכמה |
| He [= Nicomachus] said: Before Pythagoras, whoever was skilled in any craft or medicine was called "wise", in a general term, without investigating the principle by which something should be named by the term "science" | אמר וכבר היה אשר היו קודם פיתאגוריש וקראו כל מי שהיה פקח בדבר מהמלאכות או הרפואה חכם בשם משולח מבלתי שיחקרו על השורש אשר בו ראוי להקרא בשם החכמה |
| Pythagoras, reserving this name to its meaning and to the root from which it is derived, has called "science" specifically - excluding the sciences that derived from it - the true knowledge of permanent things, i.e. the species and genera. | אמנם פיתאגוריש למה שייחד זה השם לעיניינו והשרש אשר ממנו נגזר קרא אמתת הידיעה בדברים המתמידים ר"ל המינים והסוגים החכמה בפרט מבלתי מה שיצא מזה מן החכמות |
| He [= Nicomachus] said: it is appropriate to call the science "sofia" and the love of it "philosophy", i.e. the love of science. | אמר והראוי מה שיקרא החכמה סופיה והאהבה לה הפילוסופיה ר"ל אהבת החכמה |
| He said: The statement about its definition is the more accurate and it should be accepted, rather than [the one of] those who defined it by a different definition, since it was already shown that its definition is derived from its name. | אמר הנה יותר ראשון שיצדק המאמר בגדרה ויותר ראוי בשנקבל ממנו בלתי מי שגדרה בזולת זה הגדר אחרי שכבר הורנו על שגדרה נגזר משמה |
Abū Yusūf said: The ancients defined philosophy by several definitions
|
אמר אבו יוסף כי הקדמונים גדרו הפילוסופיה במספר גדרים |
|
אם מגזרת שמה כמו אוהב החכמה לפי שפילוסוף הוא מורכב מפילו והוא אוהב וסופיה והוא החכמה |
|
ואם שגדרוה מפעולתה ואמרו שהפילוסופיאה ההתדמות בבורא ית' כפי יכולת האדם ירצה בזה שיהיה האדם שלם בחשיבות |
|
וגדרוה עוד מצד פעולתה ואמרו שהיא ההשגחה במות והמות אצלם שתי מיתות |
|
וגדרו גם כן הפילוסופיא מצד העלה כשאמרו הפילוסופיא היא מלאכת המלאכות וחכמת החכמות |
|
וגדרו גם כן הפילוסופיה כשאמרו כי היא ידיעת האדם עצמו |
|
וזה מאמר נכבד ורחוק מהעול מאד |
|
ודרך משל אומר שהדברים אחר שהיו גשמים ולא גשמים והיו הגשמים עצמים והיו לא גשמים אם עצמים ואם מקרים והיה האדם הוא בעל הנפש והגשם והמקרה והיתה הנפש עצם לא גשם הנה כשידע האדם עצמו ידע הגשם במקריו והמקרה הראשון והעצם אשר הוא לא גשם וכאשר ידע זה יחד הנה כבר ידע הכל ולזאת העלה קראו החכמים האדם עולם קטן |
| [Al-Kindī] defines philosophy as the eternal universal things - their essences, their beings, their causes, and their whys, according to the human comprehension capacity. | ואמנם מה שנגדור בו אנחנו הפילוסופיה הוא שהפילוסופיה היא ידיעת הדברי' הנצחיים הכוללים ישתויותיהם ומהיותיהם ועלותיהם ולמויותיהם כפי יכולת האדם להשיגו |
| The words of Abū Yusūf are completed. | שלמו דברי אבו יוסף |
| The author of this book [= Nicomachus] said: he [Pythagoras] further defined science by saying that science is the veracious knowledge of permanent things. | אמר מניח הספר ועוד שהוא גדר החכמה בשאמר שהחכמה אמיתת הידיעה בדברים התמידים |
| He has also defined knowledge by saying that knowledge is the apprehension of the purpose of things that are ascribed to knowledge, i.e. those that are permanent, whose existence does not change, whose being does not change, and who never depart from their quality. These are the primary natural species and genera, which - when the individuals are associated and described by them - deserve the name of existing, since their species give them names and definitions. | עוד גדר הידיעה כשאמר שהידיעה היא השגת תכלית הדברים המיוחסים לידיעה ר"ל אותם התמידיים אשר לא יעתקו מעניין מציאותם ולא ישיגם שינוי בישותם ולא יותרו מתכונתם והם המינים והסוגים הראשונים בטבע אשר בהשתתפות האישים להם וכשהם מתוארים בם היו ראויים לשם הנמצא אחר שיתנו מיניהם שמותיהם וגדריהם |
| The bodily perceptible individuals are in perpetual disintegration and in continuous change. They are similar, however, by nature and property, to the first property of the primeval matter from which they were formed. By property, the intention is to the element, because the whole element was a single sphere, which has been transformed and modified. | ואמנם האישים המוחשים הגשמיים הנה הם בהתוך מתמיד ובשנוי מדובק והנה עם זה מתדמי הטבע והסגולה אל הסגולה הראשונה מן ההיולי אשר בו התוו ורצוני בסגלה היסוד כי היסוד כלו היה גלגל אחד השתנה והותר |
| Abū Yusūf said: He means that the exalted Creator has made certain qualities as certain causes. | אמר אבו יוסף ירצה בזה שהבורא ית' שם קצת תכונה לקצת עלות |
| As an example, the sight, which he made as the cause of visible colors, and the hearing, which he made as the cause of the existence of the audibles. | ודרך משל אומר כראות אשר שמו עלה לנראים מהגוונים והשמע אשר שמו עלה למציאות הנשמעים |
| Likewise, he has made the faculty, which is called the nature, as the cause of the movement of the moving bodies that are resting after the movement. | וכן שם הכח אשר יקרא הטבע עלה לתנועות המתנועעים הנחים אחר התנועה |
| The moving bodies are the bodies, and because of this the bodies are called nature, since those that receive their movement and their rest by nature are therefore always deficient and changing. | והמתנועעים הם הגשמים ולזה יקראו הגשמים טבע לפי שאשר יקבלו מהתנועה והמנוחה אמנם יקבלוהו מן הטבע הנה הם בזה בחסרון לעולם ובתמורה |
| Also the perceptible bodies, i.e. the individuals, are always affected by shifting and change. | וכן הגשמים המוחשים ר"ל האישים יקרה להם ההעתק והתמורה תמיד |
| Those of them that exist are affected by change in substance and in nature. | ואמנם אשר תחת ההויה מהם הנה התמורה תקרה להם בעצם ובטבע |
|
ואמנם הגלגל ומה שבו הנה תקרה להם ההעתקה במקום |
|
ואמנם האש והאויר והמים והארץ יקרה להם ההעתק והתמורה בקצת בלתי הכל |
| Each one of those, whose change was described, is affected by it during its whole existence. | וכל אחד מאלו אשר תארנום במה שיקרה להם מהתמורה הנה הם בו כל ימי היותם |
| By his saying "the first property of the primeval matter", he meant the individuation of the individuals, who are transformed from a non-being into a being, and "the element" is the potentiality. | ואמרו הסגלה הראשונה מן ההיולי ירצה בו איוש המתאיישים שהם יומרו מה שהיו מלא אל יש והיסוד הוא האפשרות |
| The author of this book [= Nicomachus] said: Those that are non-bodies, which are what exists, i.e. the nine categories of accidents, due to their defined separation, do not deviate from their quality, and do not change their essence, meaning, the secondary accidents, for they are apprehended together. | אמר מניח הספר ואמנם אשר אינם גשמים והם מה שימצא ר"ל מאמרות התשעה המקרים הנה הם בגדר הפרדתם בלתי סרים מתכונתם ולא משתנים מענינם ירצה המקרים השיניים כי הם מושכלים יחד |
| Yet, the change and transformation that occur in these individual perceptible accidents - such as the whiteness of such, the movement of such - they are due to their participation in the substance that carries them, i.e. when they subsist, they are affected by the change and transformation of it. | אבל מה שישיג אישי אלו המקרים המוחשים כמו לובן פלני ותנועת פלני מהשנוי והתמורה הנה הם להשתתפם לעצם הנושא אותם ר"ל כשהם עומדים ישיגם השינוי והתמורה בתמורתו ושינויו |
| Because of this, the knowledge of them is not called science. | ולזה ידיעתם לא תקרא חכמה |
| On the other hand, the secondary substances and the secondary accidents are those whose particular knowledge is the science. | אבל העצמים השניים והמקרים השניים הנה הם אשר ידיעתם בפרט היא החכמה |
| Although, the knowledge of the individuals is called science metaphorically, since it is the path towards the knowledge of those permanent, eternal and primary things, which are not subject to change nor to removal of their nature, and which are said to exist truly and distinctly. | ואמנם על ההעברה הנה תקרא ידיעת האישים חכמה אחר שהיא הדרך אל ידיעת אותם הדברים המתמדיים הנצחיים הראשונים אשר לא ישיגם שנוי ולא הסרה מטבעם אשר יאמר שהם נימצאים באמת ובברור |
| But, the individuals that are subject to the six movements, which are the generation and corruption, the growth and decline, and the change and displacement, they are said to exist because of the existence of their species, since, as explained, they give them their names and definitions. | אבל האישים הנופלים תחת התנועות השש אשר הן ההויה וההפסד והגדול והחסרון וההשתנות וההעתק הנה אמנם יאמרו שהם נימצאים מפני מציאות מיניהם אחר שמיניהם כמו שבארנו יתנו להם שמותיהם וגדריהם |
| Indeed, when we aim at one of their individuals, there is no being having its nature, since it does not exist, even for a single moment, in one and the same property, for the individuals, at any time, are in motion and in transformation. | ואמנם כאשר נכוון אל אחד מאישיהם לא ימצא בטבעו נמצא לפי שלא יתקיים כהרף עין אל עניין אחד אחר שהאישים בכל עת להם תנועה ותמורה |
| Because of this, Plato said in Timaeus: What is the thing that always exists, without ever becoming? And what is the thing that always becomes without ever being? | ולזה אמר אפלטון בספר טימאוש מה הדבר אשר הוא נמצא לעולם ואין לו התהוות כלל ומה הדבר אשר יתהווה לעולם ואין לו מציאות כלל |
| By the first, he meant the species and genera that are homogeneous eternally, which are not subject to generation, and which are indeed apprehended by the intellect, i.e. by guidance from what is perceptible. | ירצה בראשון הסוגים והמינים המתדמים לנצח אשר לא תשיגם הויה אמנם יושגו בשכל ר"ל בהדרכה מהמוחשות |
| By the second, he meant the individuals that are thought to exist at one time, but which are not really so, because they are subject to generation and transformation, without being of the same property at two different times. | ואמנם השני ירצה בו האישים אשר יחשב שהם נימצאים בעת אחד ואינם כן באמת אחר שיתהוו ויומרו מבלתי שימצאו בשני עיתים בענין אחד |
| He [= Nicomachus] said: It is appropriate for us and necessary to us, if we desire the perfection of man - as far as it is possible - and the permanent survival, that it will be achieved by philosophy alone not by anything else. | אמר הנה כבר ראוי לנו ויחוייב עלינו אם היינו נכספים לשלמות האדם כפי יכלתו וההשארות המתמיד והיה זה אמנם יהיה בפילוסופיה לבדה לא בדבר זולתה |
| Philosophy is, as we have mentioned, the love of science. | והפילוסופיה כמו שזכרנו אהבת החכמה |
| Science is the veracious knowledge of existing things. | והחכמה אמיתות הידיעה בדברים הנימצאים |
| Among the existing things, some are said to truly exist, and some are called such by homonymy. | והדברים הנימצאים מהם מה שיאמר לו נמצא באמת ומהם מה שיאמר זה בשתוף השם |
| It is necessary to examine the existing things and explain them according to the truth. | מחוייב שנחקור מהדברים הנמצאים ונבארם באמתות |
| We say that among the perceptible existences, there are those whose parts are unified, their members are integrated - this is as the rank of the animal, whose parts are unified in it, or the tree, and their like - those are called magnitudes truthfully. | ונאמר שהנמצאות המוחשות מה שהם מתאחדי החלקים מחוברי האיברים וזה כמדרגת החי אשר חלקיו מתאחדים בו וכמו כן האילן והדומה להם ואילו יאמר להם גדלים באמתות |
| And among them are those that are dissociated, so that when they are close in places and when they gather, they are called sums and multitudes - even though their parts are not unified - like the herds of goats and the rows of people. | ומהם מה שהם מפורדים והם בהתקרב מקומותיהם ובהתקבצם יקראו כללים ורבויים ואם לא יהיו מתאחדי החלקים כמו עדרי הצאן ושורות האנשים |
| It has thus been clarified that, of the perceptible things, some are subject to magnitudes and some of them are subject to plurality. | הנה כבר התבאר שמהמוחשים מהם מה שהוא נופל תחת הגודל ומהם מה שהוא נופל תחת הרבוי |
Yet, these two species are infinite by nature, generally speaking.
|
אלא ששני אלו המינים אין תכלית להם בטבע כאשר יאמרו מאמר משולח |
| For, the plurality, starting from a limited origin and multiplied, does not have by nature an end at which it would stop, and it does not multiply a single time, but it always continues to grow. | כי הרבוי כאשר יתחיל משרש מוגבל ויכפיל לא יהיה לו תכלית בטבע יעמד אצלו ולא יהיה לו כפל אחד אבל יוסיף ויצמח תמיד |
| Likewise, the magnitude, beginning its division from a limited totality, the division does not stop at an indivisible part, but always admitting division. | וכמו כן הגודל כאשר יתחיל בחלוקתו מכללות מוגבל לא תכלה החלוקה אל חלק אין חלק לו אבל יסבול החלוקה תמיד |
| Therefore, the knowledge of these two does not really exist, because the knowledge of the infinite does not exist. | הנה אין ידיעת שני אלו נמצאת על האמת אחר שידיעת מה שאין תכלית לו בלתי נמצאת |
| Indeed, true knowledge is the knowledge of circumscribed limited things. | ואמנם הידיעה על אמיתתו ידיעת הדברים הנכללים המוגבלים |
| It has thus been clarified that the knowledge does not recognize the absolute plurality, because it is infinite, and what is infinite is unlimited, and what is unlimited there is no way whatsoever to its knowledge. | הנה כבר התבאר שהידיעה לא תעמד אצל הרבוי המשולח לפי שהוא בלתי בעל תכלית ומה שהוא בלתי בעל תכלית הוא בלתי מוגבל ומה שהוא בלתי מוגבל אין דרך אל הידיעה בו כלל |
| However, if we speak of the plurality in relation to the less and we call the two related terms by their specific names, then they are knowable: | ואמנם אם נאמר הרבוי בהצטרף אל המיעוט ונקרא שני המצטרפים בשמותם המיוחדים היו אז ידועים |
|
לפי שהרב אמנם יהיה רב אצל מה שהוא פחות ממנו וכמו כן המעט אמנם הוא מעט אצל מה שהוא יותר ממנו |
|
והמעט אצל מה שהוא יותר ממנו הוא הרב אצל מה שהוא פחות ממנו |
|
כמו העשרה שהוא הרבה כשהוקש אל מה שתחתיו ומעט כשהוקש אל מה שעליו |
|
וכן הגדול אמנם הוא גדול כשהוקש אל מה שהוא יותר קטן ממנו ואם הוקש אל מה שהוא יותר גדול ממנו היה קטן |
| It has thus been clarified from these two aspects that, although they are unlimited by themselves, they are nevertheless limited when they are in relation. | הנה כבר התבאר משני אלו הצדדים שאפלו היו בלתי מוגבלים בעצמם הנה הם עם ההצטרף אליהם מוגבלים |
| Let us now say that the numerical quantity is divided into two categories: | ונאמר עתה שהכמה המספרי יחלק לשני חלקים |
|
אחד מהם הוא אשר מה שחוייב לו יחוייב לו לבדו ובטבעו |
|
והאחר הוא שמה שיחוייב לו יחוייב לו בהצטרף |
|
אמנם המחוייב לו בטבע כמו הזוג והנפרד וזוג הזוג וזוג הנפרד והנפרד הראשון בלתי המורכב והשני המורכב |
|
כי אלו אמנם יחוייבו לכמה המספרי בטבעו לא מפני הצטרפו אל זולתו |
|
כי אין הזוג מתחלק לשני חלקים מתמשלי האחדים מפני הצטרפו אל דבר אחר |
|
ואמנם אשר יחוייב לו מה שיחויב בהצטרף כמו הכפל והחצי והשליש |
|
כי החצי לדבר מה חצי והכפל לדבר מה כפל |
| It has, therefore, been clarified that the quantity is a science investigated by two arts: | הנה אם כן התבאר שהכמה חכמה יחקרוה שתי מלאכות הם |
|
הארתמאיתיקא והיא תחקור מהכמה הניפרד |
|
והמוסיקא והיא תחקור מהכמה המצורף |
| [The science of quality is investigated by two arts:]
The quality of natural things is divided into two categories: quality of the moving and quality the resting. |
ואמנם איכות הדברים הטבעיים יחלק לשני חלקים אם איכות מתנועע ואם איכות נח |
|
והמתנועע הוא מה שיחקור במלאכת האסטרונומייא ר"ל התכונה |
|
והנח הוא מה שיחקר במלאכת הגימטריא ר"ל ההנדסה |
| He [= Nicomachus] said: There is no other way to arrive at the knowledge of the species that have been said to truly exist, except through these four arts which are: arithmetic, geometry, astronomy and music. | אמר הנה אין דרך אל ידיעת מיני מה שנאמר שהם נימצאים באמת רק באלו האומניות הד' אשר הם הארתמאיטיקא וההנדסה והתכונה וחבור הנגונים |
| There is no path leading to philosophy except through them. | ולא ימצא דרך אל הפילוסופיה אלא בם |
| As, in every art, the practitioner must have the expertise in his art and needs a model which directs him in the execution of his plan, so are these sciences for the art of the philosophy, which is the art of the arts. | וכמו שכל מלאכה מן המלאכות יצטרך עושה אל בקיאות במלאכתו ודמיון יתישר ממנו בהוצאת דרושו כן אלו החכמות במלאכת הפילוסופיה אשר היא מלאכת המלאכות |
| This has already been said by Androcydes the Pythagorean. | וכבר יאמר זה המאמר אנדרוקודיס הפיתאגוריי |
| Archytas of Taranto, too, at the beginning of his Art of the Composition of Melodies, also said: The ancients did well to see from these sciences, that it is by no means impossible that they will have for everything a unique and true opinion. | וכן יאמר גם כן ארגוטאלס אל אטראנטיני כאשר התחיל במלאכת חבור הליחנים שהוא אמר הטיבו הראשונים במה שראו מענין אלו החכמות כי אינו בלתי אפשר כלל שיהיה להם בכל דבר סברה אחת אמיתית |
| Just as they excel in the nature of the whole, they were willing to have a true opinion on each of the parts. | הנה כמו שהם כבר הטיבו במה שראו מענין טבע הכל כן היו מוכנים לסבור בכל אחד מן החלקים סברא אמיתית |
| For they offered us in the sections of geometry and astronomy a clear and obvious science, and they were no less useful in giving us the virtues of the art of music. | כי הם הקנו לנו בשערי המדות והתכונה חכמה גלויה ומבוארת ולא קצרו גם כן מזה במה שהועילונו מעלות מלאכת הלחנים |
| These sciences can be imagined as four brothers. | וכבר ידומה שאלו החכמות הארבעה אחים |
| Yet, they are reducible to the first two species, namely the quantity and the quality, as explained previously. | ואם היו כבר ישובו אל שני המינים הראשונים אשר הם הכמות והאיכות כמו שביארנו לפנים |
| Similarly, Plato, at the end of the thirteenth treatise of the book he wrote on laws, which is the book that people call the "Philosophy", examining and defining what is proper for a true philosopher to be in the knowledge of these four, summarized it, after having explained it at long, saying that any shape, sum of numbers, harmonic music, and what is indicated by the movements of the planets, its knowledge should necessary be practice individually by the measure of their harmony. | עם שאפלטון בסוף האופן השלשה עשר מהספר אשר הניח בנימוסים והוא הספר אשר יקראוהו האנשים הפילוסופיא בחקרו וגדרו איך ראוי שיהיה אשר הוא פילוסוף באמת כן בידיעת אלו הארבעה אחר כן שב ואמר בקצרה אחר שהפליג בזה והקדים שכל תמונה וקיבוץ מספר והסכמת חבור ומה שיורו בו ממרוצות הכוכבים אמנם ראוי שיורגל ידיעתו כפי שעור חבורם אחד אחד |
| The cause of what is mentioned is clear: when man intensively learns to apprehend through them one known, which he expects of them, they aim at one thing, even though they are several. | וכבר יתבאר עלת מה שזכרנוהו כי כאשר היה האדם אמנם יתלמד הרבה להשיג בם ידוע אחד הוא כונתו מהם הנה אמנם הם דבר אחד ואם רבו |
| However, if man exchange this path by the science of philosophy, he will not reach it, since it is the way in which the path leading to the existence is reached, there is no other way at all, i.e. the four mentioned sciences, whether difficult or easy, numerous or few. | ואמנם אם יחליף האדם זה הדרך בחכמת הפילוסופיא אין דרך לו אליה לפי שזה האופן אשר ממנו יבא הדרך אשר ממנו המציאות אין דרך לו זולת כלל ר"ל החכמות הארבעה אשר זכרנו קשות היו או קלות רבות היו או מועטות |
| Their acquisition should not be neglectful, for who has united these sciences in the described mode, is the one whom I call a true scholar and describe as indulging in his science. | אין ראוי להתרשל בקניניהם כי מי שקיבץ אלו החכמות על צד שתארתי הוא אשר אקראהו אני חכם אמתי ואתארהו שש בחכמתו |
| It is not hidden that these sciences are like bridges through which our thoughts pass from those perceptible apprehended things to the existing intelligible things, they transfer our thoughts from those corporeal things, with which we have grown and which are familiar to us, to things which are foreign to us, which are not familiar to our senses, and which, by their subtlety, are similar to our souls. | כי לא יעלם שאלו החכמות הם כמו גשרים בם יעברו מחשבותינו מאלו הדברים המוחשים המחושבים אל הדברים הנימצאים המושכלים ויעבירו דעותנו מאלו הדברים הגשמיים אשר גדלנו בם והרגלנום אל הדברים הזרים אצלנו אשר לא הרגילום חושינו ואשר הם בדקותם דומים לנפשותינו |
| The best suited of what was chosen in the introduction to the investigation of the sciences and their acquisition is what Plato mentioned in the Book of Laws that Socrates told him, and he tries to be clever by putting forward true and useful reasons for these sciences that are necessary for the sciences that are useful for the improvement of human lives, for he said: | והיותר ראוי ממה שבחרנוהו בהקדמת העיון בחכמות וקנינם מה שזכרו אפלאטון בספר הנימוסים שסקראט אמר לו והוא יתחכם כשיביא עלות אמיתיות מועילות בענין אלו החכמות מתחייבות מצד החוכמות מועילות בתיקון חיי בני אדם אחר שיאמר |
|
אמנם מלאכת החשבון אמנם נצטרך אליה בחשבונות או בחלוקות ובתבואות ובהוצאות ופרעון החובות והשותפיות |
|
אמנם המדות בהשואת המחנות ובניין המדינות וההיכלות ומדידת השדות |
|
אמנם חכמת הכוכבים בידיעת עיתות עבודת הקרקעות ורכיבת הים וזולת זה מבחירות עיתות התחלות המלאכות ופרק הזמנים |
|
אמנם חכמת הניגונים להודות בם הבורא ית' או לשמוח במקהלות או בצניעה |
| To this Plato replied, rebuking him: do you think, seeing that I am indulgent towards you, that I will not dispute you? | ולזה יאמר אפלאטון משיב לו והוא יוכיחהו התחשבני כי תראה שאחמול עליך שלא אחלק עמך |
| I say that these sciences can not be doubted; for this dispute would be quite difficult from every side. | הנני אומר שאלו החכמות אין לדקדק בם שזה החלוק יקשה מאד מכל צד |
| But, I will add support: there is no way to say that the eye of the soul, i.e. when you are blinded and other corporeal activities are closed off, spreading a veil over you, it is opened and awakened in these sciences. | אבל אוסיפך חזוק שאין דרך לאחד לומר זה כי עין הנפש ר"ל כאשר יעצומוהו ויסתמוהו שאר הפעולות הגופיות וישימו עליך מסך הנה הוא יפקח ויעור באלו החכמות |
| The truth of the eye of the soul is more excellent than the truth of ten thousand bodily eyes, for it is only through it that the truth of things can be seen. | ואמתת עין הנפש יותר משובח מאמתת עשרת אלפים עינים מהעינים הגופים כי בו לבדו יראו אמתויות הדברים |
| The order of the natural sciences He [= Nicomachus] said: The first of these four sciences, the one which is anterior to the others by nature, the one that is best established, that is for them in the rank of the generative, from which the beginning of their existence and the root of their growth, is the art of arithmetic. |
אמר והיותר ראשונה מאלו החכמות הארבעה והיותר קודמת על הנשארות בטבע והיותר חזקת האמונה והיא להם כמדרגת המולידה אשר ממנה התחלה היותן ושרש צמיחתן הוא מלאכת החשבון |
| It is not because that it is that which will exist in the government of the Creator, but because it was, in a rank of an allegory, first in the thought of God, and the metaphor, from which are derived the properties of the things which the exalted produced from the foundation and which he has completed according to the specific properties of each. | אין זה לפי שהיא היא אשר תתקיים בהנהגת הבורא אבל לפי שהיא במדרגת ההמשל היתה ראשונה במחשבת האל והדמיון אשר ממנו יקחו תכונת הדברים אשר הצמיחם ית' מהיסוד והשלימם על הענינים המיוחדים אשר עליהם כל אחד מהם |
| The author of this book [= Nicomachus] said: what indicates that the art of arithmetic is, by nature, anterior to others, is that its disappearance would lead to the disappearance of others, while the disappearance of one of these would not lead to its disappearance. | אמר מניח הספר ויורה על מלאכת החשבון ג"כ יותר קודמת בטבע מזולתה שהיא תשים זולתה מאלו החכמות אובד באבדה מבלתי שתאבד היא באבדן דבר מהן |
| For, if the number disappears, the counted disappear; but, if they the counted disappear, the number does not disappear. | כי אם יסתלק המספר יעדרו הספורים ולא אם יעדרו הספורים יאבד המספר |
|
כאמרנו בחי שהוא יותר קודם מן האדם קדימה טבעית שכאשר יעלה החי יעלה האדם בהעלותו ולא כשיעלה האדם יעלה החי |
|
וכן כל דבר יותר קודם מדבר אחר קדימה טבעית כשיעלה הקודם יעלה בהעלותו זולתו ולא יעלה הוא בהעלות דבר ממה שהוא יותר קודם ממנו |
|
וכן כאשר תהפך המאמר תמצא הענין כפי זה כשיחויב דבר יחויב בחיובו מה שהוא יותר קודם ממנו קדימה טבעית ולא כשיחויב הקודם יהיה הכרח שיחויב בחיובו מה שהוא תחתיו בקדימה |
|
כמו האדם והמשורר כי האדם יותר קודם מהמשורר וכשיחויב משורר יחויב שיהיה אדם ואין הכרח כשיחויב אדם שיהיה בלי ספק משורר |
|
הנה כפי זה הסדר אלו החכמות הארבעה אשר זכרנו כאשר תהיה המדידה אין ספק שיהיה החשבון עמה |
|
כי כשיחויב במדות משולש ומרובע ובעל שמנה תושבות או חמש עשרה תושבות הנה כבר השתמשת בזה ממלאכת החשבון לפי שהארבעה והשלשה והשמנה והט"ו מן החשבון |
|
ולא תמצא מלאכת המדות ריקה מן החשבון והמספרים אשר יחויבו בחיובה בהכרח |
|
כי אין דרך לומר בכפל שלשה פעמים וארבעה פעמים מבלתי ידיעת השלשה והארבעה קודם בהנחה |
|
ואמנם אם תהפך המאמר תמצא השלשה והארבעה וזולתה מהמספרים כבר נתקיימו בטבע והמחשבה ואם לא היו הגדלים הנגזרי השמות מהם ידועים או נמצאים |
|
הנה כבר התבאר אם כן שהמספר יותר קודם מן המדות קדימה טבעית |
|
אחר שבסור החשבון תסור המדידה ולא יסור החשבון בסורה |
|
וכן גם כן בכמו זה הבאור יתבאר שמלאכת החשבון יותר קודמת ממלאכת הלחנים |
|
לפי שהעצם יותר קודם מההצטרפות |
|
כמו שהממון יותר קודם מרבוי הממון והעצם יותר קודם מגודל העצם |
|
והאדם יותר קודם מהכלים |
|
וזה לפי שחבור הנגונים והאותותם והסכימם אמנם הוא כשיהיה תכלית הקול אחד מהם אצל האחר |
|
וזה בהסכמה אשר בארבעה ואשר בחומש ואשר בכל שלשה פעמים ואשר בכל הארבעה פעמים |
|
וכל קבוץ יחסים אמנם נרצה ממנו מספר הנעימות אשר בו |
|
ואשר בארבעה אחד מתכליותיו אצל האחר כמוהו וכמו שלישיתו |
|
ואשר בחמשה אחד מתכליותיו אצל האחד כמהו וכמו חציו |
|
ומאשר בארבעה ואשר בחמש יהיו שני התכליות ביחס אשר בכל ואשר אחד מתכליותיו אצל האחר כפלו ואשר בכל ואשר אחד מתכליותיו אצל האחר שלשה דמיוניו |
|
לפי שתכלית אשר בכל וחמש אצל אשר בכל ביחס כל וחצי כל |
|
ואשר בארבעה ובכל אחד מתכליותיו אצל האחר כשמנה אצל השלשה |
|
ואשר בכל ארבעה פעמים הוא אשר אחד מתכליותיו אצל האחר ארבעה דמיוניו |
|
ואלו כל קבוצי החבור אין דרך לחברם והחשבון בלתי נמצא |
|
וכשימצא החשבון לא יחוייב שיהיו החבורים ולא הלחנים נמצאים |
|
וכמו כן גם כן מלאכת החשבון בזה הבאור יתבאר שהיא קודמת ממלאכת התכונה |
|
וזה גלוי אחר שבחשבון והמדות יושגו כל דרושיה |
|
וכבר יתבאר גם כן שהמדה יותר קודמת מהתכונה מצד שהתנועה אמנם תהיה אחר המנוחה |
|
ועוד שכבר יאמר שתנועות הכוכבים הם נגונים נערכים ר"ל הן בייחס חבוריי פשוט ר"ל בפשיטות יחס בעל הכפלים ויחס המוסיף חלק וכאשר היה זה כן הנה החבור הנגוני יותר הקודם מהתכונה |
|
ואמנם זריחות הכוכבים ועריבותיהם וקדריותיהם והסתרתם והראותם לא יעלם שכבר יושב במדה |
| Since the art of arithmetic is anterior to the others, what is said before is applied to it, that the discussion about it and the statements concerning it are the first in order of priority, and that is what will be done from here. | וכאשר היתה מלאכת החשבון יותר קודמת מזולתה בראוי אם כן ממנה מה שהקדמנו לפנים שהמאמר עליה ומשפטי הדבור בה יותר ראשונים בקדימה ואנחנו בזה מכאן |
| Here is my brother the completion of the introduction of this book, up to the number, as you had requested, so it is. | הנה לך אחי שלמות פתיחת זה הספר כאשר שאלת עד מקום המספר ממנו והיה כן |
| May you succeed by all that the Creator has lavished upon you, and may He, by His grace, lead you to His will. Amen. | ובכל מה שהטיב הבורא עליך מצליח והוא בחסדו יישירך לרצונו אמן |
[Absolute Quantity - Arithmetic] |
|
The Discussion on the Nature of Number |
הדבור בטבע המספר |
| All things, whose composition is established by nature, are found to be subject to a numerical order | כל הדברים שתיקן הטבע חבורם ימצאו נופלים תחת סדר המספר |
| Such as the elements, the times, the movements of the spheres, and the rest of the natural phenomena. | כמו היסודות והזמנים ותנועות השמים ושאר התקונים הטבעיים |
| By this, the number is necessary at the rank of allegory and metaphor from which the thing is understood. | הנה בזה ראוי שיהיה המספר במדרגת ההמשל והדמיון אשר ממנו יוקח הדבר |
| The exalted Creator has created it as first by nature. | וחדשו הבורא ית' ראשון בטבע |
| Indeed, the composition of the number itself has no other order, as it is, in its quality, eternal by itself, not by any other. | ואמנם חבור המספר עצמו אין סדר לו זולתו לפי שהוא בתכונתו נצחי בעצמו לא עומד בזולתו |
| But, since every compound is compounded out of different things undoubtedly and out of things that also exist, | אבל לפי שכל מחובר אמנם הוא מחובר מדברים מתחלפים בלי ספק ומדברים הם גם כן נמצאים |
| (as, the non-existent - their composition is not imaginable, | אחר שאשר אינם נמצאים אין לשער חבורם ולא אשר הם |
| and if they existed, but are not similar, it is impossible to compound them), | ואפילו היו נמצאים אלא לפי שהם בלתי דומים אי אפשר חבורם |
| it follows that the compound things are compounded out of existing different suitable things | הנה נמשך לזה שהדברים המחוברים אמנם יחוברו מדברים נמצאים מתחלפים נאותים |
|
לפי שאם לא יהיו מתחלפים הנה הם אחד לא יצטרכו אל חבור |
|
ואם לא יהיו נאותים לא יהיו מתדמים ואם לא יהיו מתדמים אלא שהם הפכיים לא יפול בם החבור |
| Thus, the number is also diverse and homogeneous like this, as it is compound. | הנה המספר יהיה גם כן מתחלף ונאות כך אחר שהוא מחובר |
| This is true, since it has two primary diverse suitable homogeneous species, i.e. the even and the odd. | וזה אמת אחר שבו שני מינים ראשונים מתחלפים נאותים דומים ר"ל הזוג והנפרד |
| For the exalted Creator has compounded them with their diversity in a complicated composition, which has no separation in it, as it will be clarified in what follows. | כי הבורא ית' חברם עם חלופם חבור מסתבך אין הפרדה בו כמו שיתבאר זה במה שיבא אחר זה |
The Discussion on the Definition of Number and its Categorization |
הדבור בגדר המספר וחלוקתו |
| The number as a figurative expression is a sum of units or a total quantity that consists of units. | המספר בהעברה מן המאמר הוא קבוץ האחדים או כלל הכמות המורכב מהאחדים |
| The number is divided in a first categorization into two parts: one of them is the even number and the other is the odd number. | והמספר יחלק חלוקה ראשונה אל שני חלקים אחד מהם הזוג והאחר הנפרד |
|
והזוג יחלק לשני חלקים שוים אין ביניהם אחדות אמצעי יעדיף בו אחד מהם על האחר |
|
והנפרד הוא אשר אין דרך אל שיחלק בשני חלקים שוים אבל בהכרח כשיחלק בשני חלקים בתכלית הקירוב מן השווי היה בין חלקיו אחדות יעדיף בו אחד משני חלקיו על האחר |
|
וזה הוא הגדר כפי מה שיראו ההמון |
| According to Pythagoras: | ואמנם כפי מאמר פיתאגוריש |
|
הנה המספר הזוג הוא אשר יחלק חלוקה יראה בה היחס המקיף בתחלת המיעוט ותחלת הרבוי |
|
ירצה ביחס המקיף בתחלת המיעוט ותחלת הרבוי יחס הכפל והחצי אחר שלא יראה אלא בחלוקת המספר הזוג ר"ל המתחלק בשני חלקים שוים |
|
אמר ואמנם הנפרד הנה הוא אשר לא יקבל זה אבל הוא יחלק בשני חלקים בלתי שוים לעולם |
| According to ancients | וכפי צד מאמר הראשונים גם כן |
|
הנה המספר הזוג הוא אשר יחלק בשני חלקים שוים ויחלק גם כן בשני חלקים בלתי שוים בתוספת והחסרון |
|
מלבד השנים מהמספר הזוג שהם כאשר נחלקו יותכו אל האחדים ולא יסבלו חלקיהם התוספת והחסרון |
|
ולא יעבור הזוג בהחלקו בשני חלקים שוים או בלתי שוים מן אחד מן המספר ר"ל אם היה אחד משני חלקיו זוג היה האחר זוג ואם היה אחד מהם נפרד היה האחר נפרד |
|
והמספר הנפרד הוא אשר איך שתחלקהו לא ישתוו חלקיו ולא ימנעו מחלקיו הזוג והנפרד יחד ר"ל שאם היה אחד מחלקיו נפרד היה האחר זוג |
| Hence, it is clarified that the parts of the odds are as close to equality as possible, when there is a unit between its two parts, by which one of them exceeds the other. | אם כן הוא מבואר שהיותר קרוב מה שיהיו חלקי הנפרד מן השווי כאשר היה בין שני חלקיו אחדות יעדיף בו אחד מהם לאחד |
| According to the definition that is called Diaulos[?], whose meaning is the knowledge of one of the two unknowns through the other. | ואמנם בגדר אשר יקרא דיאלילש וענינו ידיעת אחד משני המוסכלים באחר |
|
כי המספר הנפרד הוא המתחלף משני צדדיו לזוג באחדות אם בתוספת עליו ואם בחסרון ממנו |
|
הנה אם כן בזה הגדר לא יודע הנפרד אשר הוא מוסכל עד שיודע הזוג אשר הוא מוסכל |
|
ולא יודע הזוג אשר הוא מוסכל עד שיודע הנפרד אשר הוא מוסכל |
The Discussion on the Units and the Dominion of the Unity |
הדבור באחדים ושררת האחדות |
| Every number is half its two sides [adjacent numbers] when they are summed. | כל מספר הוא חצי שתי פאותיו כאשר יקובצו |
|
ר"ל מה שהוא מוסיף מאותו המספר באחד ופוחת ממנו באחד כאשר יקובצו |
|
וכמו כן הוא חצי שתי פאות פאותיו |
|
וחצי גם כן מה שאחר זה כאשר היה מה שיחסר מאחת משתי הפיאות כשעור מה שיוסיף באחרת |
|
וכן יהיה תמיד עד שיגיע החסרון מהפאה הקטנה אל האחדות אשר לא יוכל לעברו |
| However, the unit is accompanied by the smallest number alone, which is two. | ואמנם האחדות הנה לא ילוה לו רק הפחות במספרים אשר הוא שנים |
|
ולפי שאינו מספר שיהיו לו שתי פיאות היה חצי פאתו האחת ר"ל חצי השנים |
|
אחר שתולדת השנים מכפלו |
| Hence, it is already clarified that the unit is the cause of number in its growth. | הנה כבר התבאר שהאחדות עלת המספר בצמיחתו |
The Discussion on the Categories of the Even Number and the Property of the Even-Times-Even Number |
הדבור בחלקי הזוג וסגולתו זוג הזוג |
| The even number is divided into three categories: | המספר הזוג יחלק לשלשה חלקים |
|
אחד מהם זוג הזוג |
|
והשני זוג הנפרד |
|
והשלישי זוג הזוג והנפרד |
|
הנה אם כן שני החלקים הראשונים אשר הם זוג הזוג וזוג הנפרד נבדלים להבדל הזוג והנפרד בגדר |
|
והחלק השלישי אשר הוא זוג הזוג והנפרד כאמצעי משני הקצוות ללקחו השם מורכב משמות שני החלקים |
Even-Times-Even Number |
הנה זוג הזוג |
|
הוא מורכב מהכפלת האחד |
|
אם כן הוא אשר איפשר שיחלק בשני חלקים שוים הם חצאיו |
|
וכל חצי מהם לשני חצאים |
|
וכן חצאי חצאיו |
|
עד שיגיע אל האחד לפי שהוא מהכפלתו כמו שבארנו לפנים |
|
הנה אם כן כבר התבאר שכל אחד מחצי זוג הזוג זוג הזוג |
|
וכן חצי חצייו |
|
לפי שכל אלו להם חציים ולחצייהם חציים עד שיגיעו אל האחדות |
|
ועוד כי חלקי זוג הזוג נמצאים לעולם נגזרי השם מזוג הוא זוג הזוג והגעתם ג"כ זוג הזוג |
|
כמו שמינית ס"ד אשר הוא נגזר משמנה אשר הוא זוג הזוג והגעתו שמנה והוא זוג הזוג |
|
גם כן כמו רביעיתם הנגזר בארבעה והוא זוג הזוג והגעתו י"ו |
|
וכן חצים והוא נגזר משנים והוא זוג הזוג והגעתו ל"ב והוא זוג הזוג |
|
ולא ימצא לזוג הזוג חלק נגזר השם ממספר נפרד |
|
כמו התשיעית הנגזר מתשעה |
|
והשלישית הנגזר מג' |
|
וכבר יחשב שזה המספר אמנם נקרא זוג הזוג לפי שחלקיו נגזרי השמות ממספרים זוגות והגעתם זוגות גם כן |
|
ונמצא מסגולת זוג הזוג שחלקיו אחר שהם נגזרי השמות ממספרי זוג הזוגות שאותם הזוגות גם כן הם הגעות חלקי זוגות זוגות מן המספר אשר הם לו חלקים |
|
|
כמו החצי הנגזר מב' והגעתו ל"ב מס"ד אשר החלק מל"ב מהם ב' |
|
וכאשר הנחנו מדרגות מספרי זוג הזוג בהכפלה מהאחד כפי זה הסדר א' ב' ד' ח' י"ו ל"ב ס"ד קכ"ח |
|
היו שמנה מדרגות הראשונה א' והשמינית קכ"ח |
|
והיו להם שני אמצעיים והם ח' וי"ו |
|
|
והיה החלק מי"ו מקכ"ח ח' |
|
|
ושמינית קכ"ח י"ו |
|
|
וכמו כן החלק מל"ב מהם ד' |
|
|
ורביע קכ"ח ל"ב |
|
|
וכמו החלק מס"ד מהם ב' |
|
|
וחציים ס"ד |
|
וכמו כן היה באחדות פעם אחת קכ"ח כמו שהאחד בם קכ"ח פעם |
|
הנה כבר התבאר במספרי זוג הזוג חלקיהם נגזרי השמות ממה שבתוכם מהגעתם כפי ההכפלה אשר תארנוה לפנים |
|
וכמו כן גם כן אם יושלך ממדרגותיהם מדרגה עד שיהיה מספר מדרגותיהם נפרד |
|
ויהיה הקצה הגדול מהם ס"ד |
|
תמצא שלא יבצר דבר ממה שתארנו |
|
ויהיה שמיניתו ח' אשר הוא האמצעי |
|
|
וחלק מי"ו מהם ד' |
|
|
ורביעיתם י"ו |
|
|
והחלק מל"ב מהם ב' |
|
|
וחציים ל"ב |
|
וס"ד באחד ס"ד פעם כמו שהאחד בו ס"ד פעם |
|
ועוד יתחייב למדרגות זוג הזוג כאשר יונחו על הסדר אשר המשלנו והיו המדרגות זוג ויחויב שיהיו להם שני אמצעיים |
|
יהיה מה שיתקבץ מהכאת אחד משני האמצעיים באחר שוה למה שיתקבץ מהכאת שני הקצוות אחד מהם באחר |
|
וכן מה שילווה לשני הקצוות אחד מהם באחר |
|
ומה שילוה לנלוה |
|
עד שיגיע לנלוים לשני האמצעיים |
|
ותבחן הקש זה במדרגות אשר ספרנום שיהיה מספרם זוג והם אשר תחלתם אחד וסופם קכ"ח ותמצא כמו שאמרנו |
|
וכאשר היה מספר המדרגות נפרד היה להם אמצעי אחד |
|
ויהיה מוכה האמצעי בעצמו כהכאת שני הקצוות אחד מהם באחר |
|
וכן מה שימשך להם כמו שבארנו |
|
וכבר יתחייב למדרגת זוג הזוג שיהיו כאשר נכללו מן האחד היה הגעת כלם פחות מהקצה הגדול באחד |
|
ואם לוקח עמם הקצה הגדול היה כל זה פחות מכפל הקצה הגדול באחד |
|
וזה יתחייב למדרגות ימעטו או ירבו |
|
ומבואר מזה שמדרגות זוג הזוג כשנכללו מן האחד היה הגעתם נפרד לפי שהם פחות מהקצה הגדול אשר הוא לעולם זוג באחד |
|
והחסר מהזוג אחד הוא הנפרד |
The Discussion on the Even-Times-Odd |
הדבור בזוג הנפרד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The number that is an even-times-odd number is divided into two parts of similar units, by the nature of its type, as it is an even number, but it stops there and none of its halves can be divided again into halves. | המספר אשר הוא זוג הנפרד יחלק לשני חלקים דומי האחדים למה שתקנהו מטבע סוגו אחר שהוא זוג ושם יעמוד ולא יתחלק פעם שנית אחד מחלקיו בשני חצאים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה אם כן זוג הנפרד הוא הכפלת הנפרד פעם אחת | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כמו ששה ועשרה וארבעה עשר וי"ח וכ"ב וכ"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|
כי ששה מכפל ג' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|
ועשרה מכפל ה' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|
וי"ד מכפל ז' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|
וי"ח מכפל ט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| [The production of the even-times-odd numbers:] | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
|
הנה אם כן כשיסודרו הנפרדים הטבעיים ר"ל על משך סדר הטבע ויתחילו מתחלת הנפרדים אשר הוא ג' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כמו ג' ה' ז' ט' י"א י"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והיה תוספת הנפרד על הנפרד אשר לפניו מהם שנים שנים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד תכפול אלו הנפרדים על המשכם ותסדרם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כמו ו' י' י"ד י"ח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תוסיף כל פעם על אשר לפניו ד"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
לפי שהנפרדים הטבעיים אשר חדשו אלו הזוגות מכפלם היו נוספים שנים שנים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר נכפלו יכפל התוספת והיו ד"ד כמו שהמשלנוהו ממדרגותיהם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומבואר שהם אמנם יתחדשו על משך טבעם מהכפלת הנפרדים הטבעיים פעם אחת | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכבר יתחייב לזוג הנפרד שיהיה כל אחד מחלקיו מתחלף ההגעה לשמות גזרתו בסוג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ר"ל שאם היה החלק נגזר השם ממספר נפרד היה הגעתו זוג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם היה החלק נגזר השם ממספר זוג היה הגעתו נפרד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כמו ששה מזוג הנפרד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כי שלישיתו הנגזר מג' והוא נפרד ב' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וחציו הנגזר מב' והוא זוג ג' והוא נפרד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם כן כבר התבאר שזוג הזוג מתחלף לזוג הנפרד לפי שזוג הזוג חלקיו נגזרי השמות מסוג הגעתם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כמו ח' אשר רביעיתם ב' וחציים ד' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכבר יחשב שלזה נקרא זה המין זוג הנפרד אחר שהיו חלקיו מתחלפים בשם ובהגעה בסוג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכבר יתחייב לזוג הנפרד שלא יהיה לדבר ממנו שליש אלא למה שאתאר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה שתניח מדרגות זוג הנפרד אשר התחלתם ו' על הדמיון המתואר קודם זה והם ו' י' י"ד י"ח כ"ב כ"ו ל' ל"ד ל"ח מ"ב מ"ו נ' נ"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד נעבור שלשה מדרגות מתחלת המדרגות הנה הרביעית יש לה שליש והיא י"ח ושלישיתה ו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד תתרחק מי"ח שלשה מדרגות והרביעית יש שליש והיא ל' ושלישיתה י' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן תמיד ולא ימלטו ממך מזוג הנפרד מה שיש לו שליש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| [The production of the even-times-odd numbers from the sequence of even numbers that are divisible by 3:] | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Abū Yusūf said: | אמר אבו יוסף | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואתה כאשר תקח הזוגות אשר להם שליש מתחלתם ותסדרם ימשכו קצתם לקצת | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כמו י"ח ל' ומ"ב ונ"ד וס"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותקח שליש כל אחד מהם על המשך ותסדרם ימשכו קצתם לקצת | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כמו ו' י' י"ד י"ח כ"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תמצא שלשיותיהם על משך סדרם הם מדרגות זוג הנפרד מתחלתם על סדרם לא יבצר מהם דבר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכבר ישיג לזוג הנפרד שמדרגותיו כאשר היה מספרם זוג והיו להם שני אמצעיים היו האמצעים כמו שני הקצוות מקובצים וכמו אשר ילוה לשני הקצוות ואשר ילוה לנלוה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כמו שבארנו לפנים במדרגות זוג הזוג אלא שהענין יהיה שם בהכאה ובכאן יהיה בחבור | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם היו מספר המדרגות נפרד היה להם אמצעי אחד לבד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותהיה הכפלת האמצעי האחד ממדרגות זוג הנפרד כמו מקובץ שני הקצוות וכן מה שימשך לשני הקצוות ומה שימשך לנמשך אליהם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Hence, the difference of the even-times-even numbers and the even-times-odd numbers is already clear with regard to difference between the issue of the product and the issue of the sum of the two extremes, the single mean, or the two means. | הנה כבר התבאר הנה חלוף זוג הזוג וזוג הנפרד בהתחלף ענין ההכאה וענין החבור בשני הקצוות והאמצעי ושני האמצעיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Furthermore, the even-times-even number and the even-times-odd number differ from each other also in that the even-times-odd number alone is divisible [= its other divisors are indivisible by 2], while the only indivisible part of the even-times-even number is the smallest part, i.e. 1 [= its other divisors are divisible by 2] | וכבר יתחלף זוג הזוג לזוג הנפרד גם כן כאשר המתחלק מזוג הנפרד קצהו הגדול לבד ושהבלתי מתחלק מזוג הזוג קצהו הקטן לבד אשר הוא האחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
The Discussion on the Quality of the Even-Times-Even-Times-Odd |
הדבור בתאר זוג הזוג והנפרד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The even-times-even-times-odd number is the third species of even numbers | אמנם המספר אשר הוא זוג הזוג והנפרד והוא המין השלישי ממיני הזוג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| It is as a mean between the [other types of even numbers] and consists of them, since it adopts from each of them its property | כאמצעי להם ומורכב מהם ללוקחו מכל אחד כמו סגלתו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמנם מה שלקח מדמיון זוג הזוג שהוא יחלק לשני חלקים מתמשלי האחדים בטבע אשר הקנהו סוגו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד יחלק כל אחד משני חלקיו שנית בשני חלקים מתמשלי האחדים בטבע אשר הקנהו בהכרח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואולי יחלקו חלקיו וחלקי חלקיו פעמים רבות אלא שלא תגיע בו החלוקה אל האחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה במה שיחלק לשני חלקים מתמשלי האחדים יותר מפעם אחת ידמה לזוג הזוג ויתחלף לזוג הנפרד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ובאשר לא יכלה בחצוי אל האחד ידמה לזוג הנפרד ויתחלף לזוג הזוג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| By every property, by which it is similar to one of the two species, it differs from the other species. | הנה כל מה שידמה בו אחד משני המינים יתחלף באותו הענין למין האחר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותבחן זה במספרי זוג הזוג והנפרד כמו י"ב וכ' וכ"ד ותמצאהו כן | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The even-times-even-times-odd number has properties that the even-times-even and the even-times-odd numbers do not have; and it has properties that are shared by them as well. | וכבר ישיג מספר זוג הזוג והנפרד שימצאו לו הסגלות אשר נאמרו שלא ימצאו לזוג הזוג ולא לזוג הנפרד ושימצאו לו ג"כ אותם הסגלות אשר ימצאו אליהם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמנם אשר ישיגהו ממה שלא ישיגם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
מזה שהמתחלק מזוג הנפרד קצהו הגדול לבד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והבלתי מתחלק מזוג הזוג קצהו הקטן לבד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אבל זוג הזוג והנפרד אין המתחלק לבד קצהו הגדול ולא הבלתי מתחלק ממנו קצהו הקטן לבד אבל כבר ימצאו לו בלתי קצהו הגדול חלקים יקבלו ההחלק גם כן וימצאו לו בהכרח קודם שיכלה בו ההחלק אל האחד חלק לא יקבל חלוקה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואמנם מה שישיגהו ממה שישיגם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הוא שבו חלקים נגזרי השמות ממספר מין הגעתם דומה בזה לזוג הזוג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ובו חלקים מתחלפים הגזרה במין להגעתם ידמה בזה לזוג הנפרד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Examine this and you will find it so. | ותבחן זה ותמצאהו כן | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| [The production of the even-times-even-times-odd numbers:] | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| How this species is produced and grows: | ואמנם איך יתילד זה המין ואיך יצמח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Since it shares similarities with the even-times-even number and the even-times-odd number, it consists of what they both consist of | הנה למה שהיה לוקח הדמוי לזוג הזוג ולזוג הנפרד יודע שהוא יורכב ממה שהורכבו ממנו יחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה המספר אשר הוא זוג הנפרד אמנם יתילד מהכפלת הנפרדים הטבעיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והמספר אשר הוא זוג הזוג אמנם יתילד מהכפלת האחדים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Generating the third type, which is the even-times-even-times-odd numbers: | הנה כאשר תרצה להוליד זה המין השלישי אשר הוא זוג הזוג והנפרד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תסדר הנפרדים הטבעיים אשר התחלתם שלשה ימשכו קצתם לקצת בטור הארוך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד תסדר מדרגות זוג הזוג אשר הכפלתם מד' ר"ל בזה הצד לפי שאחר הנפרד הראשון מהנפרדים הטבעיים התחלת זוג הזוג ימשכו קצתם לקצת בטור הרחב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד תתחיל ותכה המדרגה הראשונה מאי זה משני הטורים שתרצה בכל מדרגות הטור האחר אחד אחד עוד תעמיד כל מה שיצא לך על מדרגותיו בטור שלישי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד אחר זה תשוב אל המדרגה הנמשכת למדרגה אשר הכית בכל מדרגות הטור האחר ותעשה בם גם כן כמו שעשית באשר לפניה ותכה אותה בכל מדרגות הטור האחר ותעמיד מה שיצא לך גם כן מסודר בטור רביעי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן תעשה במדרגה השלישית והרביעית או מה שהנחת מהמדרגות ותסדר זה כלו כמו שאתה מראה בזאת הצורה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכבר הוספנו ברחב לוח הולך באורך ילוה ללוח אשר בו הנפרד הטבעי העמדנו בו זוג הנפרד על סדר מדרגותיו אשר התחלתם ששה כדי להראות בזה כל מה שזכרנו מהסגולות למיני הזוג השלשה אחר שזאת הצורה תקיף עליהם יחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כי אתה כאשר תשים הקצה הקטן מזוג הנפרד ששה והקצה הגדול ממנו י"ד תמצאם מקובצים כ' ותמצא האמצעי אשר הוא י' כאשר תכפלהו כמו זה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן אם שמת הקצה הגדול י"ח תמצא שני הקצוות מקובצים כ"ד וכן שני האמצעיים והם י' וי"ד כ"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואמנם זוג הזוג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה אתה אם שמת הקצה הקטן ממנו ד' והגדול י"ו תמצא מה שיתקבץ מהכאת ד' בי"ו שוה למה שיתקבץ ממוכה האמצעי והוא ח' בעצמו והוא ס"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם שמת הקצה הגדול ל"ב תמצא כלל הכאת ד' בל"ב קכ"ח והוא שוה למה שיהיה מהכאת שני האמצעיים אחד מהם באחד והם ח' וי"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| It has been clarified from the properties of even-times-even-times-odd numbers that were explained before and from what is perceived from this diagram after the completion that: | הנה כבר התבאר ממה שבארנו לפנים מסגולות זוג הזוג וזוג הנפרד וממה שנמצאהו משיג לזאת הצורה אחרי השלמה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| [odd × even-times-even-times-odd] | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שאתה כשתכה תחלת מדרגה ממדרגות הנפרד הטבעי במדרגה הראשונה ממדרגות זוג הזוג והנפרד מאי זה מן הטורים ההולכים באורך המתחילים מן הרחב שתרצה תמצא ההגעה כבר תתרחק מאותה המדרגה שני אמצעיים והיה הוא המדרגה הרביעית מאותה המדרגה המוכה בה ממדרגות זוג הזוג והנפרד באורך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תכה המדרגה השנית ממדרגות הנפרד באותה המדרגה הראשונה ממדרגות זוג הזוג והנפרד תמצא ההגעה מתרחקת מאותה המדרגה הראשונה ממדרגת זוג הזוג והנפרד חמש אמצעיים והיא מתרחקת מההגעה הראשונה שני אמצעיים ותהיה ההגעה השנית היא המדרגה הרביעית מההגעה הראשונה באורך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכמו כן ישיג כל מדרגות הנפרד שכל מה שתרד בם מדרגה תעבור הגעת אשר לפניה בשני אמצעיים באורך כאשר היתה המדרגה המוכה בה מדרגות הנפרד ממדרגות זוג הזוג והנפרד לבדו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| [even-times-even × even-times-even-times-odd] | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואמנם אם תכה המדרגה הראשונה מזוג הזוג במדרגה הראשונה מזוג הזוג והנפרד מאי זה מהטורים ההולכים ברחב שתרצה תעבור אמצעי מהמדרגה המוכה בה מזוגות הזוגות והנפרד והיית בעברך האמצעי לוקח ברחב מכוין למה שהיית עושה בהכאת מדרגות הנפרד הנה נפלת על ההגעה אשר תהיה מהכאת המדרגה הראשונה מזוג הזוג במדרגה הא' מאי זה מטורי זוג הזוג והנפרד שתרצה ההולכים ברחב המתחילים בארך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תכה המדרגה השנית ממדרגות זוג הזוג במה שהכית בו ממדרגות זוג הזוג והנפרד ר"ל המדרגה הראשונה מזוג הזוג והנפרד תמצא ההגעה כבר נתרחקה מן המדרגה המוכה בה מזוג הזוג והנפרד שני אמצעיים והייתה המדרגה הרביעית ממנה ברחב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וגם כן אם תכה המדרגה השנית מזוג הזוג והיא ח' במדרגה השנית ברחב מזוג הזוג והנפרד והיא כ"ד תמצא ההגעה מתרחקת מכ"ד שני אמצעיים גם כן ולא תתרחק מההגעה הראשונה שני אמצעיים אבל מהמוכה בה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Therefore, it is clear that when the first term of the even-times-even numbers is multiplied by the terms of any column of the even-times-even-times-odd numbers for each descending term, the product moves away by one term breadthwise from the first product. | הנה אם כן כבר התבאר שמוכה המדרגה הראשונה ממדרגות זוג הזוג כשהוכה במדרגות טור מטורי זוג הזוג והנפרד ההולכים ברחב כל מה שירד מדרגה ירד אחרת מההגעה הראשונה לוקח ברחב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Also, when the first term of the even-times-even-times-odd numbers is multiplied by the even-times-even numbers the products are arranged successively term by term breadthwise with no intermediary term in a line starting from an even-times-even-times-odd term. | ושהמדרגה הראשונה ממדרגות טורי זוג הזוג והנפרד כאשר תוכה בכל מדרגות זוג הזוג ויצאו ההגעות ימשכו קצתם לקצת בלא אמצעי בטור אשר התחלתו היא המדרגה מזוג הזוג והנפרד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| This is the end of the discussion on the three categories of the even numbers that are evident in the categorization mentioned before. | הנה זה סוף המאמר בחלקי הזוג השלשה הנראים בחלוקה אשר זכרנו לפנים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The mentioning of the perfect, the superabundant, and the deficient even number is in another place that is more appropriate than where the author of the book mentioned it, and there it will be discussed, by the will of God. | אמנם זכירת הזוג השוה והנוסף והחסר הוא בזולת זה המקום יותר ראוי באשר זכרו מניח הספר ושם נדבר עליו ברצון השם יתע' |
The Review of the Odd Number and The Explanation of its Categories |
בזכירת המספר הנפרד ובאור חלוקותיו |
| The odd number, as said before, is that which is divided by any division into two unequal parts greater and smaller, and as its division is approaches as closely as possible to equality, one of them exceeds the other by one | המספר הנפרד כמו שאמרנו לפנים הוא אשר איך שחולק חלק בשני חלקים מתחלקים בתוספת וחסרון וכשיקרבו חלוקותיו מהשווי תכלית מה שאיפשר היה ביניהם אחד יעדיף אחד מהם על האחר |
| Its nature is divided into two types and a third type accidentally occurs | והוא יחלק בטבע לשני חלקים ויקרה לו שיהיה לו חלק שלישי |
|
ואמנם האחד מהשנים אשר בטבע יאמר לו ראשון בלתי מורכב |
|
ואמנם האחר יאמר לו שני מורכב |
|
ואמנם השלישי הנה יקרה משני אלו החלקים בהצטרף והוא שיהיה נפרד שני מורכב יצורף אל נפרד שני מורכב ויהיה כל אחד מהם אצל חבירו ראשון בלתי מורכב |
The [sum] of the terms of the natural odd number is the same as the product of the number of the terms by itself 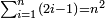 . .
|
וכבר ישיג הנפרד הטבעי שכל מספר מדרגותיו כמו הכאת מספרי המדרגות בעצמם |
| Examine it in this diagram: | ותבחן זה בצורה הזאת |
| א' ג' ה' ז' ט' י"א י"ג ט"ו י"ז י"ט כ"א כ"ג כ"ה |
The Review of the Prime Incomposite Number |
בזכירת הנפרד הראשון הבלתי מורכב |
| The first type of odd numbers is incomposite, that has no divisor but the one, such as: 3; 5; 7; 11; 13; 17. These numbers and others like them have no divisors and no fractional parts but the one which [are a part whose name] is derived from the whole number itself. | המספר הנפרד הראשון שהוא בלתי מורכב אשר אין לו חלק ימנהו בלתי האחד כמו ג' ה' ז' י"א י"ג י"ז אלו המספרים ומה שימצא כמותם לא ימצא להם מספר ימנם ואין להם חלק כלל ימנם בלתי האחדים שנגזר להם חלק מכלל המספר |
|
כמו השלישי מג' והשביעית מז' אשר הוא האחד |
| It should be called prime, because it is measured only by the unit. | הנה בראוי אם כן נאמר שהוא ראשון אחר שלא ימנהו בלתי האחדות |
| It is incomposite, since it cannot be divided into equal numbers [i.e. parts other than ones], whereas it is produced from the sequence of units. | ושהוא בלתי מורכב אחר שאי אפשר שיחלק בחלקי מספרים שוים לפי שהוא אמנם התילד מסדר האחדים והמשכם |
| An odd number has a divisor if it is a product of this type of odd number, i.e. a prime incomposite odd number. | ואמנם יהיה למספר הנפרד חלק ימנהו כאשר היה נכפל מזה המין מהנפרד ר"ל הנפרד הראשון הבלתי מורכב |
|
כי אם נכפל פעמים מספרם זוג יתילד מהם מספר הזוג |
|
והמספר הזוג לו מספר ימנהו בהכרח לפי שאפילו ימעטו חלקיו המונים אותו מאד הנה השנים ימנהו וימנהו גם כן כלל אחדי הפעמים אשר בם ימנוהו השנים |
|
ואם היו הפעמים הזוג אשר נכפל בם הנפרד שנים לבד יתחדש מכל נפרד מהם זוג הנפרד |
|
ואם נכפל הנפרד מספר פעמים והיה מספר הפעמים זוג והיה הזוג יותר משנים הנה המתחדש מהם זוג הזוג והנפרד |
|
ואם נכפל פעמים מספרם נפרד יחודש ממנו נפרד ימנהו אותו המספר הנפרד הנכפל בשעור אחדי הפעמים אשר נכפל בם וימנהו ג"כ כלל אחדי הפעמים אשר נכפל בם אותו המספר הנפרד הראשון הבלתי מורכב רצוני לומר בשעור אחדי אותו הנפרד הנכפל |
The Review of the Secondary Composite Odd Number |
בזכר המספר הנפרד השני המורכב |
| The composite odd number has a divisor beside one, and that number is a fractional part of it. | המספר הנפרד המורכב הוא אשר לו עם האחד מספר ימנהו וזה המספר הוא חלק לו |
|
וכבר אמרנו שהמספר הנפרד הראשון אמנם התילד מהמשך האחדים לבד |
|
ואמנם אם יהיה המספר נפרד נכפל הנה לא יהיה ואמנם זה הנה אמנם יצמח ויתילד מהכפלת הנפרדים הראשונים כשנכפלו פעמים מספרם נפרד |
| Hence, this type of odd number is composed of the prime odd number. | הנה זה הנפרד אם כן מורכב מהנפרד הראשון |
|
הנה הוא אם כן שני לפי שהוא אמנם חודש מהראשון |
|
והוא מורכב לפי שהנפרד הראשון חלק לו |
|
וזה כמו ט' וט"ו וכ"ה וכ"ז |
|
כי ט' מורכב מהכפלת ג' שלשה פעמים |
|
וט"ו מורכב מהכפלת ה' שלשה פעמים או מהכפלת ג' חמשה פעמים |
|
וכמו כן כ"א מהכפלת ז' שלשה פעמים או מכפל ג' שבעה פעמים |
| ? | הנה אם כן כבר יכלול זה המין מהנפרד |
| The first species [prime odd number] has a fractional part that is derived from its name, but this is not a number - it is the unity [p has the part 1/p, but this part is not a number, because it is the unit]. This is the property of the genus of genera, which is the number, i.e. that it is somposed of the units. | והמין הראשון שיש לו חלק נגזר השם משמו אינו מספר והוא האחדות כי זה סגולת סוג הסוגים אשר הוא המספר ר"ל שהוא מורכב מהאחדים |
| The secondary composite [odd number] is distinct for having a fractional part that is not derived from its name. | ואמנם מה שנתיחד בו השני המורכב הנה הוא שיש לו חלק אינו נגזר משמו ימנהו |
|
כמו ט' כי הוא ואם היה לו חלק נגזר משמו הוא האחד אשר הוא תשיעית התשעה יש לו שליש גם כן הוא השלשה |
|
וכמו כן ט"ו יש לו חומש והוא ג' ושליש ה' עם החלק מט"ו אשר הוא האחד |
The Quality of the Third Species of Odd Number - The Relatively Prime Number |
תאר המין השלישי מהמספר הנפרד והוא ראשון אצל האחר |
| It was already said in the categorization of the odd number that this type is species arises accidentally, as it is relatively that the two composite numbers have no common number that counts them [= common divisor], but each of them, regarded in its nature, has a number that counts it, which is a part [= divisor] of it. | כבר אמרנו בחלוקת המספר הנפרד שזה המין יתחדש לנפרד במקרה לפי שהוא אמנם יהיה עם ההקשה בין שני המספרים המורכבים אין להם מספר משותף ימנה אותם ולכל אחד מהם כאשר הושב אל טבעו מספר ימנהו הוא חלק לו |
|
וזה כמו ט' אשר הם מורכבים כמו שאמרנו מהכפלת ג' שלשה פעמים הנה כאשר הוקשו אל כ"ה אשר הם מורכבים מה' חמשה פעמים הנה כל אחד משני אלו המספרים אצל חבירו ראשון בלתי מורכב לפי שאין להם מספר משותף ימנם |
|
ואמנם היה שיהיה להם מספר משותף אלו היה החלק לאחד מהם חלק לאחר אחר שהחלק הוא המונה לכל |
|
ואין השלשה אשר הם חלק לתשעה חלק לחמשה ועשרים שימנום ולא החמשה אשר הם חלק לחמשה ועשרים חלק לתשעה שימנום |
|
ואמנם ישתתפו באחד אשר אינו מספר ואמנם הוא עלה למספר |
| How are these species, which are the species of the odd number, recognized, and how is each species sorted from the natural odd numbers, so that the prime incomposite is distinguished from the secondary composite? This is done by the technique that Eratosthenes called the "sieve": | אמנם איך יוכרו אלו המינים אשר הם מיני הנפרד ואיך יוברר מין מין מהם מהנפרד הטבעי עד שיודע הראשון הבלתי מורכב מהשני המורכב הנה זה בתחבולה יקראה ארסטשאש המכבר |
|
והיא שתקח הנפרדים הטבעיים אשר התחלתם ג' על סדרם הטבעי ימשכו קצתם לקצת כפי מה שבלוח אשר למעלה |
|
וכשתרצה השני המורכב מאלו הנפרדים |
|
תתחיל ותעזוב שני אמצעיים אחר המדרגה הראשונה והמדרגה הרביעית מספר שיני מורכב והמדרגה הראשונה ג' והרביעית ט' והיא שני מורכב לפי שהיא מורכבת מג' שלשה פעמים |
|
עוד עזוב ממנו שני אמצעיים והמדרגה הרביעית גם כן ממנה נפרד מורכב והיא ט"ו מורכב מה' שלשה פעמים או מג' חמשה פעמים |
|
וכן תעשה תמיד ותעזוב שני אמצעיים והרביעי מספר נפרד שני מורכב עד שתגיע אל סוף מה שהנחת מהנפרדים הטבעיים |
|
אחר תשוב תתחיל מחמשה והיא המדרגה השנית מהנפרדים הטבעיים ותעזוב אחריה ארבע אמצעיים והמדרגה הששית ממנה נפרד שני מורכב והיא ט"ו |
|
עוד המדרגה הששית מט"ו והיא כ"ה וכ"ה מורכב מהכפלת ה' חמשה פעמים |
|
עוד תעזוב ארבע אמצעיים גם כן אחר כ"ה והמדרגה הששית ממנה היא מספר נפרד שני מורכב |
|
עד שיכלה לאשר הנחת מהמספרים הנפרדים |
|
וכן תעשה כל מה שתרד מן ההתחלה מדרגה תוסיף בהשמטת שני האמצעיים שנים לכל מדרגה |
| When one does that and writes the terms of the secondary composite odds that are recognized by this technique, nothing is missing, and what remains of those that are not marked [as composite numbers] after completing this and extracting them according to the described description are the prime incomposite odd numbers. | כי כשתעשה זה ורשמת על מדרגת הנפרד השני המורכב אשר תעמד עליהם בזאת התחבולה לא יבצר ממך דבר והיה מה שישאר אחר כלותך מזה ולקיחתו כפי זה הרושם אשר תארתי לך מאשר לא יפל בם סימן הם נפרדים ראשונים בלתי מורכבים |
|
ודע שכאשר החלות במדרגה הראשונה ותעזוב שני אמצעים בין כל שני מורכב ושני מורכב |
|
תהיה המדרגה המורכבת הראשונה תצא מורכבת מן המדרגה אשר ממנה התחילה נכפלת בעצמה ר"ל בשיעור אחדיה |
|
והמדרגה השנית המורכבת מורכבת מהמדרגה הראשונה נכפלת במדרגה השנית |
|
והמדרגה השלישית המורכבת היא מורכבת מהמדרגה הראשונה נכפלת במדרגה השלישית |
|
ואם תתחיל מן המדרגה השנית ותדלג ארבע אמצעיים בין כל מורכב ומורכב יהיו המורכבים היוצאים מורכבים מהמדרגה השנית אשר החלות ממנה נכפלת בכל אחד מהאחרים |
|
וכל מדרגה ראשונה מורכבת שתצא היא מן המדרגה אשר ממנה הוחל נכפלת במדרגה הראשונה |
|
והמורכב השני מורכב מהמדרגה השנית נכפלת בעצמה |
|
והמורכב השלישי מורכב מהמדרגה השנית אשר ממנה הוחל נכפלת במדרגה השלישית מראש המדרגות והיא המדרגה השלישית מאשר ממנה הוחל |
| The "sieve" is illustrated in order to examine through it all these properties: | וכבר ציירתי לך המכבר לבחון בו כל אלו הענינים |
|
|
|
|
|
|
|
|
|
|
| ||||||||||||||||||||||||||||||||||
|
|
|
|
|
|
|
|
|
|
| ||||||||||||||||||||||||||||||||||
|
|
|
|
|
|
|
|
|
|
| ||||||||||||||||||||||||||||||||||
|
|
|
|
|
|
|
|
|
|
| ||||||||||||||||||||||||||||||||||
| Since it is already clear how to distinguish the secondary composite numbers from the prime incomposite odds by this "sieve", so that the two natural species among the species of the odd number are recognized, each of them by itself, we shall continue to the recognition of the third accidental species and discover how to distinguish, by relating two numbers, each of which is itself a secondary composite, whether they are prime each with respect to its companion, i.e. whether they have no common number that counts them [=common divisor], or, if they do have a common number that counts them, how it is found. | ואחר שכבר התבאר לך איך תברור בזה המכבר המספרים השניים המורכבים מהנפרדים הראשונים הבלתי מורכבים עד שיוכרו שני המינים הטבעיים ממיני הנפרד כל אחד מהם לבדו נמשיך לזה הכרת המין השלישי המקרי ונגלה איך יודע כשנקיש בין שני מספרים כל אחד מהם בעצמו שני מורכב אם הם עם ההקשה כל אחד מהם אצל חברו ראשון ר"ל שאין להם מספר משותף ימנם ואם היה להם מספר משותף ימנם איך נמצאהו | ||||||||||||||||||||||||||||||||||||
|
כי אנו כשנרצה לידע זה בשני מספרים הנה אנו נשליך מגדול המספרים המונחים מה שבו מדמיוני הקטן | ||||||||||||||||||||||||||||||||||||
|
וכאשר ישאר מהגדול פחות מן הקטן נשליך מן הקטן מה שבו מדמיוני הנשאר מהגדול | ||||||||||||||||||||||||||||||||||||
|
עוד נשליך מהנשאר מן הגדול מה שבו מדמיוני הנשאר מהקטן | ||||||||||||||||||||||||||||||||||||
|
עוד כן לא נסור מחסר מה שביתר מדמיוני הפחות עד שנגיע אם אל האחדות או אל שני מספרים הקטן מהם ימנה הגדול | ||||||||||||||||||||||||||||||||||||
|
ואם יגיעו אל האחדות הנה כל אחד מהם אצל האחר ראשון בלתי מורכב | ||||||||||||||||||||||||||||||||||||
|
ואם הגענו אל שני מספרים הקטן מהם ימנה הרב הנה הקטן הוא המספר המשותף אשר ימנה שני המספרים אשר הוקש אחד מהם אל האחר | ||||||||||||||||||||||||||||||||||||
|
משל זה במספרים אשר כל אחד מהם בעצמו שני מורכב ובהקש אל האחר ראשון בלתי מורכב מספר ט' וכ"ה | ||||||||||||||||||||||||||||||||||||
|
שאנו נשליך בכ"ה מדמיוני ט' וישאר ז' | ||||||||||||||||||||||||||||||||||||
|
נשליך מה שבט' מדמיוני ז' ישאר ב' | ||||||||||||||||||||||||||||||||||||
|
נשליך מה שבז' מדמיוני ב' ישאר אחד | ||||||||||||||||||||||||||||||||||||
|
והאחד ימנה השנים ואין האחד מספר | ||||||||||||||||||||||||||||||||||||
|
הנה ט' וכ"ה כל אחד מהם אצל חברו ראשון בלתי מורכב | ||||||||||||||||||||||||||||||||||||
|
ואמנם דמיון המספרים אשר כל אחד מהם שני מורכב ובהקשה ביניהם להם מספר משותף ימנם והם בו שניים מורכבים הם כמו מספר כ"א ול"ה | ||||||||||||||||||||||||||||||||||||
|
כי אנו נשליך מה שבל"ה מדמיוני כ"א וישאר י"ד | ||||||||||||||||||||||||||||||||||||
|
עוד נשליך מה שבכ"א מדמיוני י"ד וישאר ז' | ||||||||||||||||||||||||||||||||||||
|
וז' ימנה י"ד הנה כ"א ול"ה להם מספר משותף ימנה אותם והוא ז' והם בו עם ההקשה שניים מורכבים | ||||||||||||||||||||||||||||||||||||
| Therefore, it is already clear how the accidental third type is extracted from the categories of the odd number. | אם כן כבר התבאר איך יוצא המין השלישי המקרי מחלקי הנפרד | ||||||||||||||||||||||||||||||||||||
| Thus, the discussion on all the types of the number is complete. | והנה נשלם המאמר על מיני המספר יחד | ||||||||||||||||||||||||||||||||||||
| This is the diagram of the categorization of all that was discussed from the beginning of the book to this point: | וזה צורת חלוקת מה שדברנו עליו מתחלת ספרנו עד זה המקום | ||||||||||||||||||||||||||||||||||||
|
|
Second Categorization of Even Numbers |
חלוקה שנית למספר הזוג | ||||||||||||||||||||||||||||||||||||
| The even number is divided into three categories: | המספר הזוג יחלק לשלשה חלקים | ||||||||||||||||||||||||||||||||||||
|
אם נוסף ר"ל שהגעת כלל חלקיו מוסיף על כלו | ||||||||||||||||||||||||||||||||||||
|
ואם חסר ר"ל שהגעת כלל חלקיו יחסרו מכלו | ||||||||||||||||||||||||||||||||||||
|
ואם שוה ר"ל שהגעת כלל חלקיו שוה לכלו | ||||||||||||||||||||||||||||||||||||
| This third type is as a mean between the first two types, which are as separate extremes, since they are in the greatest degree of distinction in surpassing equality by addition and subtraction. | וזה המין השלישי כאמצעי בין שני המינים הראשונים אשר הם בדמות קצוות נבדלים אחר שהם בתכלית ההבדל לעברם השווי בתוספת ובחסרון | ||||||||||||||||||||||||||||||||||||
|
ונאמ' במספר הנוסף שהוא כמו הבעל חיים שאיבריו הם נוספים במקרה על האיברים הטבעיים לו ולכל אישי צורתו | ||||||||||||||||||||||||||||||||||||
|
וזה כמו י"ב וכ"ד והדומה להם | ||||||||||||||||||||||||||||||||||||
|
|
כי לי"ב חצי ושליש ורובע ושתות וחלק מי"ב ואלו החלקים מי"ב הם ששה וארבעה וג' ושנים ואחד וכלל זה י"ו והנה י"ו יותר מי"ב והם חלקיו | ||||||||||||||||||||||||||||||||||||
|
אמנם המספר החסר הנה הוא המתחלף בתאר למספר הנוסף והוא אשר הגעת חלקיו פחות מכלו | ||||||||||||||||||||||||||||||||||||
|
וידמה לזה המספר הבעל חיים שאיבריו הטבעיים חסרים משוויים הנמצא בכל אחדי צורתו בטבע | ||||||||||||||||||||||||||||||||||||
|
וזה כמו מספר ח' וי"ד והדומה להם | ||||||||||||||||||||||||||||||||||||
|
|
כי לשמנה מן החלקים חצי ורביעית ושמינית והם ד' ב' א' וכלם ז' וז' פחות מח' אשר ז' כלל חלקיו | ||||||||||||||||||||||||||||||||||||
| As it is impossible to grasp the end of the departure from equality by addition in nature, as is said in the introduction of the book, so the deficit of that which has magnitude is relatively unbounded, except by a general expression. | וכמו שהיציאה מהשווי בתוספת אי אפשר לעמד על תכליתו בטבע כמו שנאמ' בפתיחת הספר כן החסרון בכמות בעל הגודל בלתי מוגבל התכלית אחר שהוא בהצטרף אלא במאמר כולל | ||||||||||||||||||||||||||||||||||||
| The equality is one mean between two things whose end is impossible to grasp | והשווי אחד אמצעי בין שני דברים אי אפשר לעמד על תכליתם | ||||||||||||||||||||||||||||||||||||
| So is the multitude of the two types, the superabundant and the deficient, that were mentioned in the nature of the even number. | כן רבוי שני המינים אשר זכרנום מהנוסף והחסר בטבע המספר הזוג | ||||||||||||||||||||||||||||||||||||
| Yet, the perfect is rarely found, not as much as the first two species abound. | ואמנם השוה אמנם ימצא מעט לא הרבה כמו שיתרבו שני המינים הראשונים | ||||||||||||||||||||||||||||||||||||
| The perfect even number is obtained by a technique that will be reviewed now. | וזה שאנו אמנם נמצא מהמספר הזוג השוה מה שנגיע אליו לבד בתחבולה אשר נזכרה עתה בג"ה | ||||||||||||||||||||||||||||||||||||
The Discussion on the Quality of the Perfect Number and its Derivation |
הדבור בתאר המספר השוה והוציאו | ||||||||||||||||||||||||||||||||||||
| It was already said that the perfect number is that whose sum of its parts is equal to its whole. | כבר אמרנו שהמספר השוה הוא אשר הגעת כלל חלקיו שוה לכלו | ||||||||||||||||||||||||||||||||||||
| This number resembles an animal, whose organs are balanced, balanced in its form. | וזה המספר ידמה בעל חיים שוה האיברים ממוצע הצורה | ||||||||||||||||||||||||||||||||||||
|
וזה כמו מספר ו' ומספר כ"ח | ||||||||||||||||||||||||||||||||||||
|
|
כי ששה יש לו חצי ושליש ושתות והם ג' ב' א' וכלם ששה הנה אלו הששה שוים לששה אשר הם חלקיו לא יוסיפו ולא יחסרו | ||||||||||||||||||||||||||||||||||||
|
|
וכמו כן גם כן כ"ח יש לו מהחלקים חצי ורביעית ושביעית וחלק מי"ד וחלק מכ"ח והם י"ד ז' ד' ב' א' ואלו כלם שוים למספר כ"ח אשר הם כלל חלקיו לא יוסיפו ולא יחסרו מהם | ||||||||||||||||||||||||||||||||||||
| How these perfect numbers are produced, how we recognize their number and how many of them there are in every given [rank] - it is as described: | ואמנם איך יתילדו אלו המספרים השווים ואיך נעמוד על המספר הנמצא מהם וכמה מספרים ימצאו מהם בכל מספר מונח הנה הוא במה שאתאר | ||||||||||||||||||||||||||||||||||||
|
וזה שנניח מדרגות מספרי זוג הזוג אשר התחלתם מהאחד בטור | ||||||||||||||||||||||||||||||||||||
|
ותהיה המדרגה הראשונה א' והשנית ב' ונעזוב המדרגה הראשונה עוד נתחיל מב' ונניח תחתיה כלל מה שיתקבץ ממנה וממה שלפניה וזה ג' | ||||||||||||||||||||||||||||||||||||
|
וכן תעשה במה שילוה לה מן המדרגות זוג הזוג תניח תחת כל מדרגה כלל מה שיתקבץ ממנה וממה שלפניה | ||||||||||||||||||||||||||||||||||||
|
הנה בהכרח שיהיו המספרים המונחים תחת מדרגות זוג הזוג נפרדים לפי שהמדרגה הראשונה האחד והמדרגות אשר אחריה זוגות והזוג כאשר יוסף עליו האחד ישוב נפרד | ||||||||||||||||||||||||||||||||||||
|
הנה יוכרו מאלו המספרים הנפרדים כל מספר ראשון הבלתי מורכב ונרשום עליו רושם ונעזוב מה שזולתו | ||||||||||||||||||||||||||||||||||||
|
עוד נכה כל מספר שרשמנו עליו במה שלמעלה ממנו עליו ר"ל במספר הזוג אשר תחתיו אותו הנפרד הראשון הבלתי מורכב ונעמיד מה שיצא מן ההכאה תחת אותו הנפרד הראשון הבלתי מורכב כדי שיסודרו גם כן הגעות המוכים כפי מדרגותיהם בטור שלישי | ||||||||||||||||||||||||||||||||||||
| When searching by this technique all the terms of the even-times-even numbers that you set, you extract all the perfect numbers that are included in the last term, these are the numbers that are set in the third line, beneath the prime incomposite odds and they are following successively by their natural order, no perfect number among them is skipped. | וכאשר תחפש בזאת התחבולה כל מה שהנחת ממדרגות מספרי זוג הזוג הנה כבר הוצאת כל המספרים השלמים אשר הם נכללים תוך מספר המדרגה האחרונה ממה שהוצאת מהם ואותם המספרים הם אשר העמדתם בטור השלישי תחת הנפרדים הראשונים הבלתי מורכבים והם נלוים על משך סדרם בטבע לא ידולג ביניהם מספר שלם כלל | ||||||||||||||||||||||||||||||||||||
| The units that exceed the tens of the perfect numbers are necessarily 6 and 8 alternately - these numbers are always found with this quality. | וכבר יתחייב לאלו המספרים השלמים שיהיה הפרט העודף בם על עשרה פעם ו' פעם ח' וכן תמיד ימצאו אלו המספרים בזה התאר | ||||||||||||||||||||||||||||||||||||
| Examine all that was reviewed concerning their quality and their extraction method in this diagram. | ובחן כל מה שזכרתי לך מתארם ודרך הוצאתם בזאת הצורה בג"ה | ||||||||||||||||||||||||||||||||||||
|
|
The Discussion of the Quality of the Relative Quantity and its Division into the Equal and the Unequal |
הדבור בתאר הכמה הצירופיי והחלקו אל השוה ובלתי שוה |
| After elaborating the discussion on the absolute quantity, completing its discription and the explanation of its interprations, now the relative quantity will be discussed and described properly in accordance with what was described beforehand | ואחר אשר הרחבנו המאמר על הכמה הנפרד והשלמנו תאריו ובאור פירושיו אנו נקח עתה לדבר בכמה המצטרף ונתארהו תאר נאות ומסכים למה שתארנו בו מה שקדם לפניו |
| The relative quantity is divided in the first division into two parts: one is the equality, and the other is inequality. | והכמה המצטרף יחלק חלוקה ראשונה לשני חלקים אחד מהם השווי והאחר לא שווי |
| For, every number is related to a number that is either equal to it, or unequal, with no third species in this division at all. | כי כל מספר יחובר אל מספר אם שיהיה שוה לו ואם שיהיה מבלתי שוה מבלתי שיהיה בזאת החלוקה מין שלישי כלל |
| There is no supplement nor deficiency in equality, but one of the two relata is equal to the other. | הנה השווי אין תוספת בו ולא חסרון אבל יהיה בו אחד מן ב' המצטרפים שוה לאחר |
|
כמו המאה כאשר הוקשו אל המאה והעשרה אל העשרה |
|
והדומה לזה ממה שהוא שוה למה שהוקש אליו ממה שהוקשו אליו בענין הכמה אשר סגולתו שוה ולא שוה |
| The evenness is not to be divided into species at all, since the matter of evenness is the equal, the equal is equal to the equal, and the even is even to its even. | והישר לא יחלק למינים כלל לפי שאמנם ענין הישר השוה והשוה שוה לשוה והישר ישר למיושר לו |
| The species of the given numbers that differ in comparison with one another, in inequality, is divided by a second division into two parts - one of them is greater, and the other is smaller, and they are different by name and antithetical by matter. | ואמנם המספרים המונחים המתחלפים עם הצטרף קצתם אל קצת ביציאה מהשווי הנה מינם יחלק בחלוקה השנית לשני חלקים אחד מהם גדול והאחר קטן והם מתחלפים בשם ונבדלים בענין |
| The greater relation is divided in a third division into five parts: the multiple to the related to it, the superpaticular to the related to it, the superpartient to the related to it, the multiple superparticular to the related to it, and the multiple superpartient to the related to it. | וההצטרפות הגדול יחלק בחלוקה השלישית לחמשה חלקים מהם בעל הכפלים למה שהוקש אליו ומהם המוסיף חלק למה שהוקש אליו ומהם המוסיף חלקים למה שהוקש אליו ומהם בעל הכפלים המוסיף חלק למה שהוקש אליו ומהם בעל הכפלים המוסיף חלקים למה שהוקש אליו |
| The smaller is divided, like the greater related to it, into five species, each species is parallel to a species of the greater, ascribed and named according to its counterpart, i.e. the species of the smaller are called: the submultiple, the subsuperpaticular, the subsuperpartient, the submultiple-superparticular, and the submultiple-superpartient. | ואמנם הקטן יחלק כמו הגדול ההקש בו לחמשה מינים יקביל כל מין מהם מין מהגדול ייוחס ויקרא בהקבלתו ר"ל שיקראו מיני הקטן כשיאמר מין תחת בעל הכפלים ומין תחת המוסיף חלק ומין תחת המוסיף חלקים ומין תחת בעל הכפלים המוסיף חלק ומין תחת בעל הכפלים המוסיף חלקים |
[Simple Ratios] |
|
| Said Abū Yusūf: two of these five ratios are simple: the multiple ratio and the superparticular ratio | אמר אבו יוסף ומאלו היחסים החמש שנים מהם פשוטים והם בעל הכפלים והמוסיף חלק |
|
ולא ירצה במוסיף חלק בעל הכפלים המוסיף חלק אמנם רצה היחס אשר בין שני המספרים אשר הגדול מהם כמו הקטן פעם אחת וכמו חלק מהקטן |
| This is clear in what was clarified for you by Plato, in the book that is attributed to the causes of the capacities that are ascribed to the supreme individuals. | וזה מבואר במה שביארו אליך אפלטון בספר המיוחס אל עלות הכחות המיוחסות אל האישים העליונים |
|
וזה שלמה שזכר המספרים המיוחסים אל הכדורים התשעה וייחס ביניהם לחבר אל כל כדור עליון הדמוי בשכל מטבעיהם מה שיוחסו מספריהם מהמספרים המונחים לכדורי היסודות הארבעה |
|
וייחס אל כדור הארץ והמים כ"ד |
|
וייחס אל כדור האש והאויר כ"ז |
|
וייחס אל כדור הירח ל"ו |
|
וייחס אל כדור כוכב ונוגה מ"ח |
|
אחר שבאר למה היו שני אלו הכוכבים כדורם אחד והמספר המיוחס אליהם אחד בשאמר ששני אלו הכוכבים יכללם ענין אחד והוא שמרחקיהם מן השמש אמנם הוא בגלגלי הקפותיהם לבד ואמנם מרכזיהם הנה סך תנועותיהם והשמש אחד ולזה נתייחס אליהם מספר אחד |
|
ונתייחס אל כדור השמש נ"ד |
|
ולכדור מאדים ס"ד |
|
ולכדור צדק ע"ב |
|
ולכדור שבתאי צ"ו |
|
ולכדור הכוכבים הקיימים ק"ח |
|
ולא היתה הנחתו לאלו היחסים אל אלו הכדורים אשר חייבו זה המספר בהזדמן מבלתי טעם ראוי אבל אמנם נתחייב זה במאמר הכרחי המאמת שזה הוא היותר ראשון במה שיתואר מהצטרפות אלו המספרים אל אלו הכדורים בזה היחס |
|
וכאשר ייחס בין אלו המספרים זכר שק"ח מכ"ד אינו ביחס פשוט |
|
אמר ולזה נאמר שהכוכבים הקיימים רחוקים מהפעולות הגשמיות בעלות ההויה |
|
וק"ח כמו ארבעה דמיוני כ"ד וכמו חציים וזהו הכפל המוסיף חלק הנה לא יהיה זה היחס פשוט |
| This explains that he did not mean the multiple superparticular ratio by superparticular ratio. | זהו ביאור שלא רצה במוסיף חלק בעל הכפלים המוסיף חלק |
| I did not elaborate this discussion, thinking that similar discussions by the author were [not] unknown to you, in your casuistry and diligence when studying his discussion and your love to this craft, all the more so as you are among those who have his books | ולא הארכתי בזה המאמר הנה לחשבי שכמו זה ממאמרי המחבר נעלם ממך עם פילפולך ושקידתך בעיון דבריו ואהבתך לזאת המלאכה וכל שכן שאתה ממי שספריו אצלו |
| But I wanted to remind you of it. | אבל ראיתי להזכירך זה |
| I have no doubt that our book will fall into the hand of someone who is ignorant of the teacher's opinion, which you yourself know. | ולא אספק שספרנו זה יפול אצל מי שסכל מסברת המלמד מה שתדעהו אתה |
| And when the discussion has no capacity to yield the essence of the intepretation, the thoughts become confused, the conception collapses, the truths disappear, and knowledge is absent. | וכאשר לא היה בכח המאמר שיביא אל האמתת הדרוש יתבלבלו המחשבות ויפול הדמוי ויעלמו האמתיות ויעדרו הידיעות |
| May God lead you straight in the light of his explanation, to reach the brightness of his high rank. | יישירך האל לאור באורו ולהשיג זיו יקרו |
The Discussion of the Multiple Ratio - its Nature and its Production |
הדבור ביחס בעל הכפלים וטבעו והתילדו | ||||||||||||||||||||||||||||||||||||||||
| Now, we shall begin with the discussion on the multiple ratio, since it is anterior by nature to the other four remaining ratios. | ואמנם עתה נקדים המאמר על יחס בעל הכפלים אחר שהוא היותר קודם בטבע משאר היחסים הארבעה הנשארים | ||||||||||||||||||||||||||||||||||||||||
|
וזה שאנו כאשר הקשנו תחלת המספרים אשר הוא ב' אל האחד אשר הוא עלת המספר מצאנוה כפל לו וזה הוא הנקרא הכפל השניי | ||||||||||||||||||||||||||||||||||||||||
|
ואם הקשנו האחד גם כן אל המספר השני אשר הוא ג' יתחדש הכפל השלישי | ||||||||||||||||||||||||||||||||||||||||
|
ואם הקשנוהו אל המספר השלישי והוא ד' יתחדש הכפל הרביעי | ||||||||||||||||||||||||||||||||||||||||
|
עוד כן כאשר יוקש אל מדרגות המספרים הטבעיים יתחדשו כפליים יקרא בשם המספר אשר אליו יוקש האחד | ||||||||||||||||||||||||||||||||||||||||
| It is clarified that the first ratio that subsists in number is the double ratio, when the beginning of the numbers, which is 2, relates to the one, whose duplication generates the number. | הנה התבאר שהיחס הראשון שיפל במספר הוא יחס הכפלים בהצטרף תחלת המספרים אשר הוא ב' אל האחד אשר מהכפלו יהיה המספר | ||||||||||||||||||||||||||||||||||||||||
| It is clarified also that the double ratio is the first of the multiple ratios by nature, as it arises by relating the one to the beginning of the numbers. | והתבאר שהכפל השניי ראשון למיני הרב הכפליים בטבע לפי שהוא מהקשת האחד אל תחלת המספרים | ||||||||||||||||||||||||||||||||||||||||
| The species of the multiple ratios are endless in potentia, as they abound with the abundence of the natural numbers. | ואין למיני הכפלים תכלית בכח להתרבותם עם התרבות המספרים הטבעיים | ||||||||||||||||||||||||||||||||||||||||
| We will start first with the description of the production of the multiples, setting aside the other of the ratios | ונתחיל ראשונה בספור התילד בעל הכפלים בלתי שאר היחסים | ||||||||||||||||||||||||||||||||||||||||
|
ונקדים ממנו הראשון מהם בטבע | ||||||||||||||||||||||||||||||||||||||||
|
ונאמר שהכפל השניי הוא אשר יתחדש מיחס כפל כל מספר אליו | ||||||||||||||||||||||||||||||||||||||||
|
כמו ד' אצל ב' וכמו ח' אצל ד' | ||||||||||||||||||||||||||||||||||||||||
|
הנה אם כן כבר התבאר איך יצמח הכפל השניי | ||||||||||||||||||||||||||||||||||||||||
|
וזה כשיסודרו המספרים על משך סדר הטבע | ||||||||||||||||||||||||||||||||||||||||
|
כמו א' ב' ג' ד' ה' ו' ז' עד מה שנרצה להניח | ||||||||||||||||||||||||||||||||||||||||
|
עוד נסדר הזוגות הטבעיים על משך סדר הטבע בטור נכחיי לטור הראשון | ||||||||||||||||||||||||||||||||||||||||
|
כמו ב' ד' ו' ח' י' י"ב י"ד | ||||||||||||||||||||||||||||||||||||||||
|
ויהיו מדרגותיו כמספר המדרגות אשר סדרת אותם בטור סדר המספרים הטבעיים | ||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||
|
עוד תקיש הראשון ממדרגות הזוג הטבעי בראשון ממדרגות המספר הטבעי והשני בשני והשלישי בשלישי וכן במה שאחר זה שאנו נמצאם יחד ביחס הכפל השני | ||||||||||||||||||||||||||||||||||||||||
|
כמו ד' אצל ב' וו' אצל ג' וח' אצל ד' וכן כלם | ||||||||||||||||||||||||||||||||||||||||
|
אמנם הכפל השלישי הוא שיהיה בגדול משני המספרים המונחים שלשה דמיוני המספר הקטן | ||||||||||||||||||||||||||||||||||||||||
|
אם כן הוא מבואר לך איך יתילד הכפל השלישי | ||||||||||||||||||||||||||||||||||||||||
|
וזה כשתסדר המספרים כטבעיים על משך סדר הטבע בטור | ||||||||||||||||||||||||||||||||||||||||
|
כמו א' ב' ג' ד' ה' ו' ז' ח' ט' | ||||||||||||||||||||||||||||||||||||||||
|
עוד נתחיל ונעבור שני מדרגות ונקח השלישית ונשימה ראש מדרגות טור אחד | ||||||||||||||||||||||||||||||||||||||||
|
עוד נעבור גם כן שתי מדרגות אחר אותה המדרגה תקח המדרגה השלישית ונשימה ראש מדרגות השנית מהטור האחר | ||||||||||||||||||||||||||||||||||||||||
|
עוד כן נדלג שתי מדרגות אחר כל מדרגה שנקח ונחזיק בשלישית ממנה ונסדרם במדרגות אשר בטור האחר | ||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||
|
ואחר נקיש בין המדרגות מהטור המוצא ובין המדרגה הראשונה מטור המספר הטבעיי עוד השנית בשנית והשלישית בשלישית הנה אנו נמצאם ביחס הכפל השלישי | ||||||||||||||||||||||||||||||||||||||||
|
כמו ג' אצל א' ו' אצל ב' וט' אצל ג' וכן תמיד עד סוף שהנחת מן המספרים | ||||||||||||||||||||||||||||||||||||||||
|
הנה אם כן כבר התבאר שאמנם יתחדש יחס הכפל השלישיי מהכאת כל מדרגה ממדרגות המספרים הטבעיים בשלשה והעמדת אלו ההגעות במדרגות טור אחר | ||||||||||||||||||||||||||||||||||||||||
|
וכבר יתחייב לטור אשר בו מדרגות המספרים הגדולים מזה היחס ובכל יחס נגזר ההכפלה ממספר נפרד אל מספר שיהיה נפרד ואחר כן זוג עוד נפרד כפי מה שתראה אותך הבחינה | ||||||||||||||||||||||||||||||||||||||||
|
וכבר יתחייב גם כן בזה היחס לבד שיהיה בין כל נפרד ונפרד שני אמצעיים מן הנפרד הטבעי כבר עברו | ||||||||||||||||||||||||||||||||||||||||
|
כמו ה' וז' אשר כבר עברו מהנפרד הטבעי בין ג' וט' | ||||||||||||||||||||||||||||||||||||||||
|
וכן יחוייב גם כן שיהיה בין הזוג שני אמצעיים כבר עברו מהזוג הטבעי | ||||||||||||||||||||||||||||||||||||||||
|
והזוג כמו ח' וי' אשר כבר עברו בין ו' וי"ב וכמו י"ד וי"ו בין י"ב וי"ח | ||||||||||||||||||||||||||||||||||||||||
|
אמנם הכפל הרביעיי אמנם יתחדש מהכאת כל מדרגה ממדרגות המספר הטבעי בד' עוד יוקיש מה שיצא מן ההכאה אל אותה המדרגה | ||||||||||||||||||||||||||||||||||||||||
|
וכן החמשיי | ||||||||||||||||||||||||||||||||||||||||
|
ומה שאחריו ממה שתרצה ממיני הכפלים | ||||||||||||||||||||||||||||||||||||||||
| Yet, if the multiple is named by an even number, all of its terms are even numbers. | אלא שכאשר היה הכפל נקרא למספר הזוג היו כל מדרגותיו זוגות | ||||||||||||||||||||||||||||||||||||||||
|
כמו הכפל הרבעיי אשר תחלת מדרגותיו ארבעה עוד ח' י"ב י"ו | ||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||
| If the multiple is named by an odd number, its terms are an even number then an odd number. | ואם היה הכפל נקרא למספר נפרד היו מדרגותיו אחת זוג ואחרת נפרד | ||||||||||||||||||||||||||||||||||||||||
|
כמו הכפל החמשיי אשר תחלת מדרגותיו ה' עוד י' עוד ט"ו עוד כ' | ||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||
| This species is named by the greater a multiple ratio and by the smaller a submultiple ratio. | וראוי שיקרא זה המין מן הגדול בעל הכפלים ומהקטן תחת בעל הכפלים |
The Discussion on the Second Simple Ratio which is the Superparticular Ratio |
הדבור ביחס השני הפשוט והוא יחס המוסיף חלק |
| After having discussed the multiple ratio, because it is simpler and anterior by nature as explained, its natural successor, which is the superparticular ratio, is discussed: | ואחר שכבר הבאנו הדבור על יחס הכפלים אחר שהוא יותר פשוט ויותר קודם בטבע כמו שבארנו נאמ' על אשר ימשך לו המשכות טבעי והוא המוסיף חלק |
When comparing the beginning of the numbers, which is 2, with its successor, which is 3, the three is to two as itself and its half.[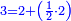 ] ]
|
וזה שאנו כאשר הקשנו בין תחלת המספרים אשר הוא ב' ובין המספר אשר ימשך לו והוא ג' היה ג' אצל ב' כמהו וכמו חציו |
| Hence, the superparticular ratio is formed by comparing the beginning of the numbers with its successor. | הנה יתחדש המוסיף חלק מההקשה בין תחלת המספרים ואשר ימשך לו |
|
והתבאר שהחלק הראשון בטבע הוא המוסיף חצי |
|
עוד המוסיף שליש בהקשת המספר השלישי אשר הוא ד' אל המספר השני אשר הוא ג' כי ד' כמו ג' וכמו שלישיתו |
|
עוד המוסיף רביע |
|
עוד המוסיף חומש |
| the ratio of each number to its predecessor among the natural numbers | בהקשת כל מספר אל אשר לפניו מהמספרים הטבעיים |
|
הנה אם כן יחוייב שנזכיר המוסיף חלק חצי |
|
ונאמר שזה היחס יתילד בשנסדר הזוג הטבעי אשר התחלתו ב' והוא הטור אשר נעשה בכפל השניי בטור ימשך קצתו לקצת |
|
עוד נסדר המספרים אשר מהכאת מדרגות המספרים הטבעיי' כל א' בג' והוא אשר נראה בכפל השלישיי בטור ימשכו קצתם לקצת |
|
עוד יוקש בין כל מדרגה מזה הטור ובין דומה לה מהטור האחר במספר המדרגות כי יראו המדרגות יחס המוסיף חלק החציי כמו שתראה בזאת הצורה |
| לב | ל | כח | כו | כד | כב | כ | יח | יו | יד | יב | י | ח | ו | ד | ב |
| מח | מה | מב | לט | לו | לג | ל | כז | כד | כא | יח | טו | יב | ט | ו | ג |
| 32 | 30 | 28 | 26 | 24 | 22 | 20 | 18 | 16 | 14 | 12 | 10 | 8 | 6 | 4 | 2 |
| 48 | 45 | 42 | 39 | 36 | 33 | 30 | 27 | 24 | 21 | 18 | 15 | 12 | 9 | 6 | 3 |
|
ואמנם המוסיף שליש הוא אמנם יתחדש בסדור זה הטור אשר מהכאת מדרגות המספרים הטבעיים בג' נכחיי לסדר הטור אשר יתחדש מהכאת המספרים הטבעיים בד' והוא הטור אשר חודש בכפל הרבעיי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכמו כן המוסיף רביע כי אחד מטוריו טור הכפל הרבעיי והאחר טור החמשיי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The same for any superparticular ratio desired, according to the example described. | וכמו כן כל מה שתרצה מהמוסיף חלק הוא כמו זה המשל אשר תארתי לך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The greater related term in this species is called superparticular and the smaller subsuperparticular. | וכבר יתכן שיקרא המוקש בזה המין אם הגדול המוסיף חלק ואם הקטן תחת המוסיף חלק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| So, for any species the smaller related term is called "sub" the specific name of the greater related term above it. | וכמו כן יקרא המוקש הקטן מכל מין כאשר הוא תחת מה שלמעלה ממנו מההצטרפות הגדול הנקרא בשם מיוחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The discussed multiple and superparticular ratios and their proper enunciation are also noted in this diagram: | וכבר רשמתי מה שזכרתי לך מהכפלים והמוסיף חלק ומה שיאות לומר גם בזאת הצורה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה שאנו נתחיל ונסדר בטור הראשון הלוקח ברחב המספרים על סדר הטבע מא' עד עשרה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ובטור השני הכפל השני נלוה לטור הראשון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ובטור השלישי הכפל השלישי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ובטור הרביעי הכפל הרביעי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן עשרה טורים ויהיה הכפל העשריי סופם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
It is clear in this figure that:
|
וגלוי בזאת הצורה ששני הטורים אשר יפגשו על מרובע א' ויתרחבו עד שטח א'ק' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ושני הטורים אשר יפגשו על מרובע ד' ויתרחבו עד שטח א'ק' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ושני הטורים אשר יפגשו על מרובע ט' ויתרחבו עד שטח א'ק' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Their ratio is one, i.e. the ratio of each term to its precedecessor in either of the two lines that meet on one of the squares that are on the diagonal, i.e. the main diagonal of the whole figure, is as the ratio of its corresponding term on the other line to its respective predecessor. | יחסם אחד ר"ל ששעור כל מדרגה מאשר לפניה מאחד מכל שני טורים מהם יפגשו על מרובע מן המרובעים אשר על הקוטר ר"ל קוטר הצורה הגדולה כשעור המדרגה אשר היא דומה לה מהטור האחר מאשר היא גם כן לפניה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Except that:
|
אלא ששני הטורים הראשונים תוסיף כל מדרגה מהם על אשר ילוה לה מכל אחד משני הטורים אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ושני הטורים השניים תוסיף כל אחת ממדרגותיהם על אשר תלוה לה שנים שנים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ושני הטורים השלישיים תוסיף כל אחת ממדרגותיהם על אשר תלוה שלשה שלשה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והרביעיים ארבעה ארבעה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The ratios of the terms of these lines, [namely] the ratio of any desired term to its predecessor, is one and the same, as they are similar. | ויחסי המדרגות מאלו הטורים יחס כל מדרגה אל אשר לפניה שתרצה אחד אחר שיהיו דומים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
For example:
|
משל זה שיחס מ"ב מהטור השביעי הלוקח ברחב אל ל"ו אשר לפניה מזה הטור כיחס ז' מהטור הראשון הלוקח ברחב אל ו' אשר לפניו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועוד יחס ז' מהטור הראשון הלוקח באורך אל ו' אשר לפניו בזה הטור כיחס מ"ב מהטור הז' הלוקח באורך אל ל"ו אשר לפניו מאותו הטור | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
לפי שהטור אשר יצא מו' מהטור הראשון ההולך ברחב הולך באורך והטור אשר יצא מו' מהטור הראשון ההולך באורך הולך ברחב יפגשו על מרובע ל"ו והיה ל"ו לכל אחת משתי המדרגות אשר בכל אחת מהן מ"ב משני הטורים המתחלפים מדרגה ראשונה לפי ששני הטורים יתחתכו על מרובע ל"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן כל שני טורים מתחלפים באורך וברחב יפגשו על מרובע מן המרובעים אשר על קוטר המרובע הגדול הנה המרובע אשר יפגשו עליו משותף להם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Hence, it is already clarified how the multiple ratio is produced from its related term: | הנה אם כן כבר התבאר איך יתילד בעל הכפלים למה שיוקש אליו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
לפי שהטור השני מטורי אי זה משני צדי האורך והרחב שתרצה אל הטור הראשון מאותו הצד כפל שניי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והשלישי אליו שלשיי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והרביעי אליו רבעיי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| So on always by oeder of the natural succession. | וכן תמיד כפי סדר על משך הטבע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| This is the explanation of the multiple ratios in this diagram. | וזה הוא המאמר על הכפלים בזאת הצורה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| As for the superparticular ratio: | אמנם המוסיף חלק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה שני הטורים שלוקח אחד מהם באורך והאחר ברחב וכבר יצאו משני מרובעי ל' ל' ויפגשו על מרובע ט' כאשר יוחסו מדרגותיהם אל מדרגות שני הטורים הלוקחים באורך והרחב וכבר יצאו משני מרובעי כ' כ' ויפגשו על מרובע ד' כל מדרגה אל אשר ילוה לה תמצא זה ביחס המוסיף חלק החציי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן יחס שאר מדרגות אלו הטורים הארבעה אל התחלותיהם ר"ל אל שני בתי ב' וג' משני הטורים הלוקחים באורך ואל שני בתי ב' וג' משני הטורים הלוקחים ברחב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואמנם יחס המוסיף חלק השלישי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה שני הטורים שלוקח אחד מהם באורך והאחר ברחב וכבר יצאו משני מרובעי מ' מ' ויפגשו על מרובע י"ו כאשר יוחסו מדרגותיהם אל מדרגות שני הטורים הלוקחים באורך והרחב היוצאים משני מרובעי ל' ל' ויפגשו על מרובע ט' כל מדרגה אשר לפניה מהטור האחר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תמצא זה ביחס המוסיף חלק השלישי בכמו אותו הביאור אשר זכרנו כמוסיף חלק החציי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן המוסיף חלק הרביעי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן המוסיף חלק החמשיי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The species of the superparticular ratio that follow in this art, derive their names from the sequence of the natural numbers that produced from the addition of the units. | ומה שאחר זה ממיני המוסיף חלק תמצאהו בזאת המלאכה נמשך בגזרת שמו ממשך המספרים הטבעיים המתילדים מהוספת האחדים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Hence it is already clarified to the perception that the most anterior among these ratios, by nature rather than by will and assumption, is the multiple ratio, due to what was ascribed to it. | הנה אם כן כבר התבאר לחוש מהיותר קודם באלו היחסים בטבע לא ברצון והנחה בעל הכפלים למה שיוחס אליו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| And the most anterior among [the multiple ratios] is the double ratio, then the triple ratio, and so on always according to the descript derivation of the numbers as they are in the natural order. | ושהקודם יותר בזה השניי עוד השלישי וכן תמיד כפי תואר הגזר המספרים כמו שהם בסדר הטבע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The superparticular ratio then follows the multiple ratio | ושאשר ימשך לבעל הכפלים הוא המוסיף חלק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| And the beginning of the [superparticular ratios] is the sesquialter ratio, then the sesquitertian ratio, then the sesquiquartan ratio and so on always according to the derivation of the numbers as they are in the natural order. | ושתחלת זה הוא החציי עוד השלישי ועוד הרביעי עוד כן תמיד כפי הגזרה מהמספרים כמו שהם בסדר הטבע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
What can be apprehended from this diagram is that the terms of the numbers on the main diagonal that goes out from 1 to its opposite, 100, each one is rooted, i.e. has an expressible root, which when multiplied by itself, i.e. by the measure of its units, it is equal to the total. [ ] ]
|
וממה שישיג סדר זאת הצורה שמדרגות המספרים אשר על קוטר היוצא מא' אל ק' הכתוב נגדו כל אחת נגזרת ר"ל שיש לו שורש וידובר בו בשיכפל בעצמו ר"ל בשעור אחדיו היה שוה לכלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כמו א' ד' ט' י"ו כ"ה ל"ו מ"ט ס"ד פ"א ק' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כי כל אחד מאלו מספר נגזר בפעל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
מלבד האחד שהוא נגזר בכח וגדרו אחד לפי שהוא אחד בעצמו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|
וגדר ד' ב' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|
וגדר ט' ג' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If the roots of these terms are taken and arranged in a line, they are found in the order of their terms, by the order of the sequence of the natural numbers, such as 1, 2, 3, 4, 5, 6, until the end of the rooted numbers that were set on the main diagonal of the square. | ואם לקחת גדרי אלו המדרגות ותסדרם בטור תמצאם בסדר מדרגותיהם על סדר משך המספרים הטבעיים כמו א' ב' ג' ד' ה' ו' עד תכלית מה שהנחת מן המספרים הנגדרים באלכסון המרובע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Abū Yusūf said: we have found these rooted numbers produced and grow from the sum of the natural odd numbers to each other by their successive order, beginning from the one, which is an odd number in potantia. | אמר אבו יוסף מצאנו אלו המספרים הנגדרים אמנם יתחדשו ויצמחו מתוספת הנפרדים הטבעיים על משך סדרם אשר התחלתם מהאחד אשר הוא נפרד בכח על קצתם על קצת | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|
ג' כאשר נוסף על האחד יתקבץ מספר נגדר והוא ד' וגדרו ב' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|
וכאשר נוסף על ד' הנפרד אשר ימשך לג' והוא ה' יתקבץ מספר נגדר והוא ט' וגדרו ג' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|
וכאשר נוסף הנפרד אשר ימשך לה' והוא ז' על ט' יתקבץ מספר נגדר והוא י"ו ושרשו ד' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן תמיד הנה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Thus, it is clarified that when the natural odd numbers, beginning from the one, which is odd in potentia, are added to each other by their successive order, the natural rooted numbers are produced by their successive order. | אם כן כבר התבאר שכאשר נוספו הנפרדים הטבעיים אשר התחלתם האחד אשר הוא נפרד בכח קצתם על קצת על משך סדרם יתילדו המספרים הנגדרים הטבעיים על משך סדרם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Their roots that are extracted from them are by their successive order as the natural numbers | והיו שרשיהם לקוחים מהם על משך סדרם על המספר הטבעי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The terms of the two secondary diagonals that follow the main diagonal are heteromecic, i.e. the two numbers that the term is a product of their multiplication one by the other - one of them exceeds the other by one, as they are conceived as close to the root of the term that has an expressible root. | ואמנם שני הטורים אשר ימשכו וילוו לקוטר הנה מדרגותיהם זולתיות האורך ר"ל ששני המספרים אשר התקבצה המדרגה מהכאת אחד מהם באחר יוסיף אחד מהם על האחר באחד בשיודמו שכבר קרבו מגדר המדרגה אשר היה להם גדר מספר ידובר בו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|
והמספרים הזולתיים כמו ו' כי הוא מהכאת ב' בג' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|
וכמו י"ב שהוא מהכאת ג' בד' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| So they are always found in the two secondary diagonals that are on both sides of the main diagonal. | עוד כן נמצאם תמיד בשני הטורים אשר משני צדי הקוטר כלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
What can be apprehended from this diagram is that the two lines that go out from the square of 1, lengthwise and breadthwise - their terms are added one by one until each of them reaches to ten that is the end of all their terms. [ ] ]
|
וממה שישיג זאת הצורה ששני הטורים היוצאים ממרובע א' באורך והרחב יתוספו מדרגותיהם אחד אחד עד שיגיע כל אחד מהם אל העשרה אשר הם סוף כל המדרגות בם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
And that the two lines that go out from the squares [i.e. cells] of 10 and meet on the square of 100 - their terms are added ten by ten. [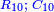 ] ]
|
וששני הטורים היוצאים משני מרובעי י"י ויפגשו על מרובע ק' יתוספו מדרגותיהם י' י' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Furthermore, if what is on the four angles of this diagram is summed, one finds this sum a rooted number. | ואם קובץ מה שבזויות זאת הצורה הארבעה תמצא כלל זה המספר נגדר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| What can be apprehended from this diagram is that the sum of the terms of each square whose main diagonal differs from the main diagonal of the entire diagram, is rooted | וממה שישיג זאת הצורה שכלל מדרגות כל מרובע יהיה קטרו נבדל מקוטר הצורה הוא נגדר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Abū Yusūf said: what is apprehended concerning the other main diagonal, whose ends are ten, and the number of its terms is even, is that it has two means, which are 30. | אמר אבו יוסף וממה שישיג הקוטר האחר אשר תכליותיו עשרה עשרה ומספר מדרגותיו זוג שיש לו שני אמצעיים והם ל' ל' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The terms that are between one of the two ends and one of the two means, the ratio between them, and the values of their numbers are in the same ratio of what is between the other end and the other mean. | והמדרגות אשר בין אחד משני התכליות עד אחד משני האמצעיים ביחס קצתם אל קצת והגעות מספריהם כיחס מה שבין התכלית האחר והאמצעי האחר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The philosopher, the author of this book [= Nicomachus] said: That which takes place in this diagram is found when its investigation is precise, for it has more beneficial and pure things than was described, but they will not be investigated in this introduction, as there was no intention to investigate them here. | אמר הפילוסוף מניח הספר כבר נמצא המקויים בזאת הצורה כאשר ידוקדק העיון בה כי בה דברים מהתועלות והזכיות יותר מאשר תארנו אלא שאנו לא נחקרם בזה המבוא לפי שאין כונתנו בו לחקרם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| It is necessary to move forward to the explanation of what is firmly agreed upon, concerning the account on the five ratios that we started discussing | וראוי שנעתק אל המאמר על מה שהוא חזק ההאותות למה שהחלונו לדבר בו מזכר היחסים החמש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Now, it should be said that the discussion of the superpartient should be anterior, as it is simpler than the two remaining ratios: | ונאמר עתה שהמוסיף חלקים למה שהוקש אליו יותר ראשון להקדים המאמר עליו לפי שהוא יותר פשוט משני היחסים הנשארים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה שבעל הכפלים המוסיף חלק הוא מורכב משני יחסים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ובעל הכפלים המוסיף חלקים מורכב גם כן משני יחסים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Furthermore, when the beginning of the odds, which is 3, is added to the second odd number, which is 5, the superbipartient is generated, which is the beginning of the species of the superpartient ratios. | ועוד שאנו כאשר חברנו ראש הנפרדים אשר הוא ג' אל הנפרד השני אשר הוא ה' יתחדש המוסיף שני חלקים והוא תחלת מיני המוסיף חלקים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Therefore, it is necessary to place the discussion of this ratio after what was said about the two first simple ratios. | ולזה יחוייב שנשים המאמר על זה היחס נמשך למה שאמרנו משני היחסים הראשונים הפשוטים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| In accordance with the natural order, the multiple superparticular ratio appears before the superpartient ratio. | ואמנם בהצטרף על סדר הטבע הנה הכפל המוסיף חלק יראה קודם מהמוסיף חלקים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה כשנקיש תחלה שני מספרים ממדרגות המספרים הטבעיים יתחדש בהקשתם יחס שלישי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והוא כמו צרוף ה' אל ב' כי ה' כמו כפל ב' וכמו חציו וחציו חלק ממנו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וה' ב' ראשוני שני מספרים ממדרגות המספרים הטבעיים יתחדש בהצטרף אחד מהם אל האחר יחס שלישי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Yet, it is clear and obvious that the superpartient ratio is simpler than the multiple superparticular ratio. | אבל הוא מבואר נגלה שיחס המוסיף חלקים יותר פשוט מיחס הכפל המוסיף חלק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The simple is anterior to the compound by natural precedence. | והפשוט יותר קודם מהמורכב קדימה טבעית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Hence, it is necessary to preface the discussion on the superpartient. | הנה אם כן יחוייב שנקדים המאמר על המוסיף חלקים אל מה שיוקש אליו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| And among its species, the superbipartient is first, since it is the first of its species. | ונקדים ממיניו המוסיף שני חלקים אחר שהוא ראש מיניו |
The Discussion of the Third Ratio which is the Superpartient Ratio |
הדבור ביחס השלישי והוא היחס המוסיף חלקים |
| Nicomachus said: there is no superpartient ratio less than the superbipartient ratio | אמר ניקומאכוש לא יהיה היחס המוסיף חלקים בפחות מהמוסיף שני חלקים |
| Hence, this ratio is formed from the sequence of the natural numbers beginning with three in a line. | ולזה אמנם יתילד זה היחס מסדר המספרים הטבעיים אשר התחלתם השלשה בטור |
| Because the number prior to three has no two parts that are smaller than it, since its parts are equal to it.[?] | לפי שהמספר אשר קודם השלשה אין לו שני חלקים יהיו פחות ממנו אחר שחלקיו שוים לו |
| He said: the terms of the natural odd numbers are arranged beneath the terms of the mentioned line, beginning with the successor of 3, which is 5. | אמר ויסודר תחת מדרגות הטור הנזכר מדרגות הנפרדים הטבעיים אשר הראשון מהם ימשך לג' והוא ה' |
| Then, each term is related to the one above it. | עוד נקיש בין כל מדרגה ואשר למעלה ממנו |
| Thus, all successive species of the superpartient ratio are revealed, according to the rule of their succession in nature. | ויראו לנו כל מיני המוסיף חלקים נמשכים כפי משפט המשכם בטבע |
| If one wishes to see the production of each species of this ratio: | ואמנם כאשר תרצה שתראה איך צמיחת כל מין ממיני זה היחס |
|
הנה עתה תעמיד שני המספרים הראשונים הפועלים למין ההוא במדרגה אחת ותניח הקטן למעלה מהגדול |
|
עוד תכה כל אחד מהם בב' ותעמיד שני המספרים המגיעים אחר ההכאה במדרגה תמשך לשני המספרים הראשונים כל אחת מהן תמשך לדומה לה הגדול ימשך לגדול והקטן ימשך לקטן |
|
עוד תשוב אל שני המספרים הראשונים ותכה אותה גם כן בג' ותעמידם במדרגה שלישית על הצד אשר העמדת בו שני המספרים במדרגה השנית |
|
וכן גם כן תכה שני המספרים הראשונים בד' ותעמידם כפי הגדר הנזכר במדרגה הנגזרת השם מהמספר אשר הכית שני המספרים הראשונים בו |
| The same is done always when wishing to yield any species of the superpartient ratio. | וכן תעשה תמיד כאשר תרצה להצמיח מין ממיני המוסיף חלקים |
| Setting up the first numbers that produce each species of the superpartient ratio by the order of these natural species, so that no species of them would fall in the vacuum of the given numbers - it is as mentioned in the introduction of this chapter, and as the first numbers that produce the species of the superpartient ratio that are illustrated below. | ואמנם איך תניח המספרים הראשונים הפועלים למיני המוסיף חלקים על סדר אותם המינים הטבעיים עד שלא יפול מהם מין בפנוי מה שיונח מהמספרים הנה הוא כמו שנזכר בפתיחת זה הפרק וכמו שכבר המשלנו תחת זה המספרים הראשונים הפועלים למיני יחס המוסיף חלקים |
| This is the diagram: | וזאת הצורה ועד כאן דברי מחבר הספר |
| יט | יח | יז | יו | טו | יד | יג | יב | יא | י | ט | ח | ז | ו | ה | ד | ג |
| לז | לה | לג | לא | כט | כז | כה | כג | כא | יט | יז | טו | יג | יא | ט | ז | ה |
| 19 | 18 | 17 | 16 | 15 | 14 | 13 | 12 | 11 | 10 | 9 | 8 | 7 | 6 | 5 | 4 | 3 |
| 37 | 35 | 33 | 31 | 29 | 27 | 25 | 23 | 21 | 19 | 17 | 15 | 13 | 11 | 9 | 7 | 5 |
superbitertian ratio
|
מוסיף ב' שלישיות
| ||||||||||||||||||||||||||||||||||
supertriquartan ratio
|
מוסיף ג' רביעיות
| ||||||||||||||||||||||||||||||||||
| Until here the author's words. | ועד כאן דברי מחבר הספר | ||||||||||||||||||||||||||||||||||
| How the other species are extracted, which are similar to whichever of these first species that one wishes: | אמנם איך יוצאו שאר המינים הדומים באי זה מין שנרצה מאלו המינים הראשונים | ||||||||||||||||||||||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
|
כמו הדומה דרך משל במוסיף שני שלישים תחת המוסיף שני חומשים ושני שביעים ושאר מה שיפל תחת המוסיף שני חלקים | ||||||||||||||||||||||||||||||||||
|
או במתדמים לנפלם תחת המוסיפים שלשה חלקים | ||||||||||||||||||||||||||||||||||
|
או בלתי שני אלו מאי זה ממיני המוסיף חלקים | ||||||||||||||||||||||||||||||||||
| The obvious way for finding this is by arranging the natural numbers in a line, such that the first of its terms is the number that is the number of the additional parts of the species intended to be added to its extracted similar. | הנה הדרך הגלוי אל מציאות זה הוא בסדור המספרים הטבעיים בטור יהיה ראש מדרגותיו המספר אשר הוא מספר החלקים הנוספים במין המכוון להוסיף להוצאת הדומים לו | ||||||||||||||||||||||||||||||||||
|
ותנשא המדרגה הראשונה על השנית ויונח מה שיתקבץ תחת השנית | ||||||||||||||||||||||||||||||||||
|
עוד נניח המדרגה הראשונה גם כן עם השלישית תחת השלישית | ||||||||||||||||||||||||||||||||||
|
וכן תונח הראשונה עם הרביעית תחת הרביעית | ||||||||||||||||||||||||||||||||||
|
ונעשה זה תמיד עד תכלית המונח מן המספרים הטבעיים | ||||||||||||||||||||||||||||||||||
|
עוד תקיש בין כל מדרגה ממנה והדומה לה | ||||||||||||||||||||||||||||||||||
| An example is given of the superquadripartient ratio, in order that the mathematician will examine it and will be led straight by it in other [cases]. | וכבר הנחתי לזה משל מהמוסיף ארבעה כדי שיבחנהו החושב ויתישר בו בזולתו | ||||||||||||||||||||||||||||||||||
| There are species of superpartient ratio that are included in other species of superpartient ratio: | ודע שמהמוסיף חלקים מה שיכנס במין אחר מהמוסיף חלקים | ||||||||||||||||||||||||||||||||||
|
כמו ו' וי' כי הם בהצטרף המוסיף הארבעה שתויות | ||||||||||||||||||||||||||||||||||
|
והם גם כן בהצטרף המוסיף שני שלישים | ||||||||||||||||||||||||||||||||||
| There are species that are included in a ratio of another genus i.e. the superparticular ratio: | וממנו גם כן מה שיכנס בזולת סוגו ר"ל במוסיף חלק | ||||||||||||||||||||||||||||||||||
|
כמו ח' וי"ב שהם בהצטרף המוסיף הארבעה שמיניות | ||||||||||||||||||||||||||||||||||
|
והם גם כן בצרוף המוסיף חלק ר"ל חצי | ||||||||||||||||||||||||||||||||||
| If one wishes to know the absolute ratios that are not included in another species, al-Kindī's effort to set a special technique for each species is not enough, since he strove for what is impossible to complete. | ואם תרצה במין מה להכיר הצרופים הגמורים אשר לא יכנסו בזולת מינם לא יספיק בזה מה שטרח בו אלכנדי מהניח מלאכה מיוחדת לכל מין לפי שהוא השתדל במה שלא נוכל לבא עד תכליתו | ||||||||||||||||||||||||||||||||||
| However, the general technique for all that was brought of this is to skip each term of the terms of the natural numbers, whose number is commensurable with the number of the additional parts. | אבל המלאכה הכוללת לכל מה שהובא מזה היא שנדלג ממדרגות המספרים הטבעיים כל מדרגה שיהיה מספרה משותף למנין החלקים הנוספים | ||||||||||||||||||||||||||||||||||
| The meaning of commensurability is that one of the two numbers is part of the other, or else, only if they have a common divisor other than one that counts both of them. | ור"ל בהשתתפות שיהיה אחד משני המספרים חלק לאחר ולא יהיה זה אלא אם להם חלק משותף בלתי האחד ימנם יחד | ||||||||||||||||||||||||||||||||||
| Its explanation was already introduced in the account on the even number and the account on the composite odd number. | וזה כבר עבר ביאורו בזכירת המספר הזוג וזכירת המספר הנפרד המורכב | ||||||||||||||||||||||||||||||||||
| Here, a technique is required for recognizing the terms that should be skipped. | ומכאן תבוקש התחבולה בהכרת המדרגות אשר נצטרך הנה לדלגם | ||||||||||||||||||||||||||||||||||
| These, as was said, are the terms: either such that ratios of the numbers of the additional parts to their corresponding numbers are in a superparticular ratio, or else, if the numbers of the additional parts are not a part of their corresponding numbers, such that their numbers and the number of the additional parts have a common part that counts them both other than one. | והיה כמו שאמרנו המדרגות אשר אם שיהיה מנין החלקים המוסיפים מכל אחד ממספריהם כי צירופי אלו יכנסו במוסיף חלק ואם שלא יהיה מנין החלקים המוסיפים חלק מכל אחד ממספריהם אלו שמספריהם ולמנין החלקים הנוספים חלק משותף להם בלתי האחד ימנה אותם יחד | ||||||||||||||||||||||||||||||||||
| For, these ratios, even if they are not included in any other than their genus, i.e. in the superparticular ratio, they are included in a species other than their species within the superpartient ratio. | כי צירופי אלו ואם לא יכנסו בבלתי סוגם ר"ל במוסיף חלק הנה יכנסו במין בלתי מינם מהמוסיף חלקים | ||||||||||||||||||||||||||||||||||
| Hence, when the terms that are commensurable to the number of the parts are skipped, the terms, whose ratios are not included in another genus, remain, and they are the absolute of the terms of the superpartient ratio. | הנה כאשר תדלג אלו המדרגות המשותפות למנין החלקים הנה כבר השארת המדרגות אשר לא יכנסו צרופיהם בבלתי מינם והם הגמורים ממדרגות המוסיף חלקים | ||||||||||||||||||||||||||||||||||
| We describe the above example by this diagram, with the marking of the commensurable terms: | וכבר תארנו משל זה שאמרנו בזאת הצורה עם רושם השותפים הנזכרים בה |
| שתוף | שתוף | שתוף | שתוף | שתוף | שתוף | שתוף | שתוף | שתוף | שתוף | שתוף | שתוף | |||||||||||||
| כח | כז | כו | כה | כד | כג | כב | כא | כ | יט | יח | יז | יו | טו | יד | יג | יב | יא | י | ט | ח | ז | ו | ה | ד |
| לב | לא | ל | כט | כח | כז | כו | כה | כד | כג | כב | כא | כ | יט | יח | יז | יו | טו | יד | יג | יב | יא | י | ט |
| common | common | common | common | common | common | common | common | common | common | common | common | |||||||||||||
| 28 | 27 | 26 | 25 | 24 | 23 | 22 | 21 | 20 | 19 | 18 | 17 | 16 | 15 | 14 | 13 | 12 | 11 | 10 | 9 | 8 | 7 | 6 | 5 | 4 |
| 32 | 31 | 30 | 29 | 28 | 27 | 26 | 25 | 24 | 23 | 22 | 21 | 20 | 19 | 18 | 17 | 16 | 15 | 14 | 13 | 12 | 11 | 10 | 9 |
[Compound Ratios] |
|
The Discussion on the Fourth Ratio which is the Multiple Superparticular Ratio |
הדבור ביחס הרביעי והוא יחס הכפל המוסיף חלק |
| The author of this book [= Nicomachus] said: The simple species among the species of the relative quantity are the species of the three ratios of the preceding discussion. | אמר מניח הספר אמנם המינים הפשוטים ממיני הכמה המצטרף הנה הם מיני שלשת היחסים אשר קדם המאמר עליהם |
| For they are like causes and principles for the two remaining species, i.e. the multiple superparticular ratio and the multiple superpartient ratio. | כי הם כהתחלות וכפנות לשני המינים הנשארים ר"ל מין הכפל המוסיף חלק מן הכפלים המוסיף חלקים |
| Since these two species are produced from the first species and are absorbed in them. | אחר ששני אלו המינים כבר יתילדו מהמינים הראשונים ויותכו אליהם |
| The simple has also another aspect, by which the species of the multiple ratio and the superparticular ratio are set aside from the superpartient ratio, in that the first is said to resemble to the second. | ולפשוט גם כן צד אחר ייוחד בו מין הכפל ומין המוסיף חלק בלתי מין המוסיף חלקים והוא בשיאמר שהוא יתדמה הראשון ממנו לשני |
| It is said to be simple, since its meaning is that the two numbers that relate in each of these two species are assimilated one to the other: | ויאמר לו פשוט לפי שעניינו אמנם הוא של שני המספרים המצטרפים בכל אחד מאותם שני המינים התדמות בעצמם |
|
אם התדמות שני המספרים במין הכפל באשר הגדול מחובר מהקטן |
|
ואם התדמות שני המספרים במין המוסיף חלק באשר הם יחד מחוברים מפנה אחת והוא המותר אשר ביניהם |
| Therefore, the ancients related the natural things by relation of these two species alone, and did not relate them by the third species that is said to be simple as well, i.e. the superpartient ratio. | ולזה הקישו הראשונים הדברים הטבעיים החבור בשני אלו המינים לבד ולא יקישו אותם במין השלישי אשר יאמר לו שהוא פשוט גם כן ר"ל במין המוסיף חלקים |
| The two compound species: | ואמנם שני המינים המורכבים |
|
הנה אחד מהם מורכב מבעל הכפלים ומהמוסיף חלק |
|
ואמנם האחר מורכב מבעל הכפלים ומהמוסיף חלקים |
| Since the part is anterior by nature to the parts, the multiple superparticular ratio is anterior to the multiple superpartient ratio. | ולפי שהחלק קודם בטבע על החלקים היה הכפל המוסיף חלק קודם על הכפל המוסיף חלקים |
| Therefore, it is necessary to begin with the discussion of the multiple superparticular ratio. | ולזה יחוייב להקדים המאמר על הכפל המוסיף חלק |
| It is said that this ratio is conceived of two numbers, one of them is greater by once or many times the other and its part. | ונאמר שזה היחס יראה משני מספרים אחד מהם יותר לאחר מדמיון אחד או דמיונים הרבה וכמו חלק ממנו |
|
כמו החמשה כאשר יוקשו אל שנים כי החמשה שני דמיוני שנים וכמו חציים |
| This species corresponds the two species of which it consists: | וכבר יקח זה המין הדמוי משני המינים אשר הוא מורכב מהם |
|
אמנם מה שיקח מיחס הכפל הנה ברבות כפלו והחלק אחד בלתי מומר |
|
כמו הכפל השניי המוסיף חצי והכפל השלישיי המוסיף חצי והכפל הרביעיי המוסיף חצי |
|
וכן תמיד ברבות הכפלים והחלק אחד לא יומר |
|
ואמנם מה שיקחהו מדמיון המוסיף חלק יומרו חלקיו ולא יתרבו כפליו |
|
כמו הכפל השניי המוסיף חצי והכפל השניי המוסיף שליש והכפל השניי המוסיף רביע והכפל השניי המוסיף חומש |
|
עוד כן יומרו חלקיו ולא יתרבו כפליו |
|
וכבר יתחדש לו והתרכבו מהם קבוץ שני סגולותיהם כאשר יתרבו כפליו ויומרו חלקיו |
|
כמו הכפל השניי המוסיף חצי והכפל השלישי המוסיף שליש והכפל הרביעיי המוסיף רביע |
|
וכן תמיד יתרבו כפליו ויומרו חלקיו |
| Forming the species according to the superparticular ratio: | ואמנם יתחדש המין האחד לדמוי מהמוסיף חלק לבד |
|---|---|
|
באשר תסדר המספרים הטבעיים אשר התחלתם ב' בטור |
|
עוד תסדר הנפרדים הטבעיים אשר התחלתם ה' בטור |
|
ונקיש כל מדרגה אל הדומה לה כמו שתראה בזה הדמיון בזאת הצורה צורת הדמיון שנזכרנו |
| כז | כו | כה | כד | כג | כב | כא | כ | יט | יח | יז | יו | טו | יד | יג | יב | יא | י | ט | ח | ז | ו | ה | ד | ג | ב |
| נה | נג | נא | מט | מז | מה | מג | מא | לט | לז | לה | לג | לא | כט | כז | כה | כג | כא | יט | יז | טו | יג | יא | ט | ז | ה |
| 27 | 26 | 25 | 24 | 23 | 22 | 21 | 20 | 19 | 18 | 17 | 16 | 15 | 14 | 13 | 12 | 11 | 10 | 9 | 8 | 7 | 6 | 5 | 4 | 3 | 2 |
| 55 | 53 | 51 | 49 | 47 | 45 | 43 | 41 | 39 | 37 | 35 | 33 | 31 | 29 | 27 | 25 | 23 | 21 | 19 | 17 | 15 | 13 | 11 | 9 | 7 | 5 |
| Finding the species according to the multiple ratio: | ותמצא המין האחד לדמוי מהכפל לבד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
|
כשתסדר טור באורך יהיה ראשיתו שני המספרים הפועלים ביחס הכפל המוסיף חלק והם ב' ותחתיו ה' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד תסדר תחת הה' מה שימשך לו ממדרגות הנפרד הטבעי ימשכו קצתם לקצת יורדים ותשים אלו המדרגות יתחילו בטורים לוקחים ברחב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
יתוספו מדרגות כל טור מהם כמנין המדרגה הראשונה ממנו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד תקיש כל אחת ממדרגות זה המרובע באשר נכח לו והוא מקבילו מהטור הראשון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שאתה תמצא בזה כבר העמדת בו יחס הכפל מבלתי שיצמח החלק וזה כמו שתראה בכאן | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Forming the species according to the two principles of which it consists | ויתחדש זה המין האחד ליחס משני פנותיו אשר הוא מורכב מהם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| That [species] whose multiples increase and whose parts are changed. | והוא אשר יתרבו כפליו ויומרו חלקיו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
באשר תסדר הנפרדים הטבעיים אשר התחלתם מה' בטור הנרשם באודם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולא נרצה להקיש בו אבל לשומו רושם לקחת דמיון ממנו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה שאנו נסדר טור אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
יהיה ראש מדרגותיו גם כן ה' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והמדרגה השנית כמו אשר לפניה ר"ל כמו ה' מוסף עליו מה שלמעלה ממנו ר"ל מהטור הראשון האדום אשר לפניה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והמדרגה השלישית כמו אשר לפניה ר"ל כמו י' מוסף עליו מה שהוא למעלה ממנו ר"ל המדרגה השנית מהטור האדום | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן תעשה עד שיכלה אל סוף מה שהנחת מהמספרים בטור האדום | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר הולדנו זה הטור השני כן סדרנו נכחו טור המספרים הטבעיים והתחלתו מב' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד נקיש בין מדרגותיו ובין מדרגות הטור אשר הולדנו ונעזוב הטור האדום | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| By relating them it is apparent how the species that corresponds to its two sides is produced - this is the third species of the multiple superparticular ratios. Examine it in the diagram we made and it will lead you straight: | ויראה בהקשתם איך יתילד המין הלוקח הדמוי משני פאותיו וזה המין השלישי ממיני הכפל המוסיף חלק ותבחנהו בצורה שעשינו והתישר בו |
| נט | נז | נה | נג | נא | מט | מז | מה | מג | מא | לט | לז | לה | לג | לא | כט | כז | כה | כג | כא | יט | יז | טו | יג | יא | ט | ז | ה | |
| תתקא | תתמב | תשפה | תשל | תרעז | תרכו | תקעז | תקל | תפה | תמב | תא | שסב | שכה | רצ | רנז | רכו | קצז | קע | קמה | קכב | קא | פב | סה | נ | לז | כו | יז | י | ה |
| ל | כט | כח | כז | כו | כה | כד | כג | כב | כא | כ | יט | יח | יז | יו | טו | יד | יג | יב | יא | י | ט | ח | ז | ו | ה | ד | ג |
| 59 | 57 | 55 | 53 | 51 | 49 | 47 | 45 | 43 | 41 | 39 | 37 | 35 | 33 | 31 | 29 | 27 | 25 | 23 | 21 | 19 | 17 | 15 | 13 | 11 | 9 | 7 | 5 | |
| 901 | 842 | 785 | 730 | 677 | 626 | 577 | 530 | 485 | 442 | 401 | 362 | 325 | 290 | 257 | 226 | 197 | 170 | 145 | 122 | 101 | 82 | 65 | 50 | 37 | 26 | 17 | 10 | 5 |
| 30 | 29 | 28 | 27 | 26 | 25 | 24 | 23 | 22 | 21 | 20 | 19 | 18 | 17 | 16 | 15 | 14 | 13 | 12 | 11 | 10 | 9 | 8 | 7 | 6 | 5 | 4 | 3 |
The Discussion on the Fifth Ratio which is the Multiple Superpartient Ratio |
הדבור ביחס החמישי והוא יחס הכפלים המוסיף חלקים |
| As was said, it is composed of the multiple ratio and the superpartient ratio. | הוא כמו שאמרנו מורכב מיחס הכפל ומיחס המוסיף חלקים |
| Therefore, the technique of the production of this ratio is divided into three parts: | ולזה גם כן כבר תחלק המלאכה בהולדת זה היחס לשלשה חלקים |
|
אם שתשתדל בם להראות סגולת הכפל ברבות כפליו מבלתי השתנות החלקים הנוספים או תמורתם |
|
ואם שתתחכם בם להראות סגולת המוסיף חלקים בהמרת החלקים בלתי רבות הכפלים |
|
ואם שיראו בם שתי הסגולות יחס הראות נמשך כמו שהוא בטבע |
| The species whose multiples increase while its parts do not change is created when placing the two initial numbers that produce whichever multiple superpartient ratio one wishes: | הנה המין אשר יצמחו כפליו ולא ישתנו חלקיו יתחדש כשנניח שני המספרים הראשונים הפועלים ליחס אי זה הכפל המוסיף חלקים שנרצה |
| לז | לה | לג | לא | כט | כז | כה | כג | כא | יט | יז | טו | יג | יא | ט | ז | ה | |
| שסב | שכה | רצ | רנז | רכו | קצז | קע | קמה | קכב | קא | פב | סה | נ | לז | כו | יז | י | ה |
| יט | יח | יז | יו | טו | יד | יג | יב | יא | י | ט | ח | ז | ו | ה | ד | ג | ב |
| 37 | 35 | 33 | 31 | 29 | 27 | 25 | 23 | 21 | 19 | 17 | 15 | 13 | 11 | 9 | 7 | 5 | |
| 362 | 325 | 290 | 257 | 226 | 197 | 170 | 145 | 122 | 101 | 82 | 65 | 50 | 37 | 26 | 17 | 10 | 5 |
| 19 | 18 | 17 | 16 | 15 | 14 | 13 | 12 | 11 | 10 | 9 | 8 | 7 | 6 | 5 | 4 | 3 | 2 |
| For example: the multiple superbitertian ratio | וכאלו נרצה יחס הכפל המוסיף שני שלישים |
|
הנה נניח שני המספרים הראשונים הפועלים להם והם ג' וח' |
|
וכאשר נרצה שאר הכפלים המוסיפים שני שלישים נעזוב הקטן על ענינו ונסדר תחתיו טור באורך לוקח תחת ח' יתחילו מדרגותיו מח' יתוספו במניין אחרי המספר הקטן ר"ל שיתוספו ג' ג' |
|
עוד נקיש כלם במדרגה הראשונה ר"ל במספר הקטן אשר הוא ג' כי הם יהיו אליו ביחס הכפל המוסיף אותם החלקים הראשונים |
|
וכבר התילדו הכפלים כפי המשכם בטבע מבלתי שיתוספו החלקים או יומרו |
| By this technique whichever multiple superpartient ratio one wishes is produced. | ובזאת המלאכה יתילדו איזה מן הכפלים המוסיף חלקים שתרצה |
| Such as: the multiple supertriquartan ratio, or the multiple supertriquintan ratio, or whichever one wishes of this. | כמו הכפל המוסיף שלשה רביעיות או שלשה חמשיות או מה שתרצה מזה |
| You will be well guided in it in the example given here. | ותתישר בו במשל אשר המשלתיו לך הנה |
| The production of the species whose parts change and multiply by a natural successive reproduction according to the increase of number from which their names are derived [= the denominator], while the number of its multiples does not change: | ואמנם איך יתילד המין אשר יומרו חלקיו ויעתקו ברבות המספר אשר ממנו יגזרו שמותיהם העתק נמשך בטבע מבלתי שיומר מנין כפליו |
| Its technique is divided also into two parts | זה גם כן תחלק מלאכתו לשני חלקים |
|
לפי שאנו אם שנלך בזה אל תולדת המינים הראשונים מהמוסיף חלקים כפי המשכם בטבע |
|
כמו המוסיף שני חלקים עוד המוסיף שלשה חלקים עוד המוסיף ארבעה חלקים עוד מה שילווה לזה |
|
ואם שנלך אל הולדת מין אחת מהם בהוצאת כל יחס יפל תחת שם אותו המין |
|
כמו המוסיף שני חלקים לבד והמוסיף שלשה חלקים לבד |
| The technique that leads to the first species of the superpartient ratio according to their natural succession, without increasing the multiples: | אבל המלאכה אשר תביא אל המינים הראשונים מהמוסיף חלקים כפי המשכם בטבע מבלתי שירבו הכפלים |
|
אמנם היא כשנסדר המספרים הטבעיים שהתחלתם ג' בטור |
|
ותניח תחת ג' המספר אשר יפעל עמם תחלת יחס המוסיף חלקים |
|
עוד נשלים הטור הגדול במדרגות יתוספו ג' ג' |
|
פעם א' אם רצינו שיהיה הכפל שניי |
|
ואם רצינו אותו שלישיי כזה שני פעמים |
|
ואם רצינו אותו רביעיי שלשה פעמים |
|
וכן מה שאחר זה |
|
עוד נקיש כל מדרגה באשר למעלה ממנה ר"ל הדומה לה מהטור הראשון |
| Thus, the multiple superpartient ratio is revealed according to the natural production of the first species of the superpartient ratio, without increasing the multiples. | הנה יראה לנו יחס הכפל המוסיף חלקים כפי התילד המינים הראשונים מהמוסיף חלק בטבע בלתי שיתרבו הכפלים |
| As seen in this example - examine it and you will find it with God's help. | וזה כפי מה שתראה בזה המשל ותבחנהו ותמצאהו בע"ה |
| יח | יז | יו | טו | יד | יג | יב | יא | י | ט | ח | ז | ו | ה | ד | ||
| נג | נ | מז | מד | מא | לח | לה | לב | כט | כו | כג | כ | יז | יד | יא | ח | ג |
| 18 | 17 | 16 | 15 | 14 | 13 | 12 | 11 | 10 | 9 | 8 | 7 | 6 | 5 | 4 | ||
| 53 | 50 | 47 | 44 | 41 | 38 | 35 | 32 | 29 | 26 | 23 | 20 | 17 | 14 | 11 | 8 | 3 |
| The production of the species of the multiple superbipartient for example, or the species of the multiple supertripartient, or the species of the multiple superquadripartient, or others, without increasing the multiples and without changing the number of the parts, but changing the number from which they are derived according to natural growth: | ואמנם איך יתילדו מיני הכפלים המוסיפים שני חלקים דרך משל או מיני הכפל המוסיף שלשה חלקים או מיני המוסיף ארבעת חלקים או זולת אלו מבלתי שיתרבו הכפלים ולא ישתנה מנין החלקים אבל ישתנה בצמיחה הטבעית המנין אשר הם נגזרים ממנו |
|
וזה כשנוציא המתדמים בכפלם תחת המוסיף אותם החלקים כמו שכבר גלינו בשער המוסיף חלקים |
|
עוד ינשא המספר הקטן מכל מדרגה על הגדול אשר נכחו אם תרצה שיהיה הכפל השניי |
|
ואם תרצה שיהיה שלישיי תשא כפל הקטן |
|
ואם תרצה אותו רבעיי תשא שלשה כפלי הקטן |
|
וכן מה שאחר זה בכל מדרגות הטור הגדול |
|
עוד נקיש כל מדרגה בנכחית לה |
| The superpartient ratio of these parts is found without increasing the multiples or multiplying the desired number. | שאתה תמצא המוסיף אותם החלקים בלתי שיוסיפו הכפלים או יעתקו מהמנין אשר תרצה |
| Examine what was mentioned in both descriptions in the chaper on the superpartient ratio, which are the species of the superpartient ratio whose number does not change, and you will be well guided by them in the production of this species of the multiple superpartient ratio by observing carefully what was noted. | ובחן מה שזכרתיו לך בשני הרשמים לפניך בשער המוסיף חלקים והם טור מיני המוסיף חלקים לא יומר מנינם ותתישר מהם להולדת זה המין מהכפל המוסיף חלקים כאשר תטיב להתבונן במה שזכרנוהו |
| The third type of the first division mentioned in the beginning of this chapter - the production of the species whose multiples increase and whose parts change: | ואמנם המין השלישי מהחלוקה הראשונה הנזכרת בפתיחת זה הספר והוא איך יתילדו המינים אשר יצמחו כפליהם וישתנו חלקיהם |
| Since the change of the parts is either by the constancy of their number and the natural increase of their values, or by the increase of their number and the increase of their values together, the technique of extracting them is necessarily divided into two parts: | הנה לפי שהשתנות החלקים אם שיהיה בקיום מנינם והתרבות הגעותיהם התרבות טבעי ואם שיהיה בהתרבות מנינם והתרבות הגעותיהם יחד יחוייב שתחלק המלאכה בהוצאת זה לשני חלקים |
|
אבל יתילד המין אשר יתקיים בו מניין החלקים וישתנו הגעותיהם בהתרבות הטבעיי |
|
תהיה מלאכתו כאשר נוציא שני טורים מיחס המוסיף חלקים מנינם ר"ל מנין החלקים הנוספים כמניין אשר תרצה לחייבו |
|
ותתישר בזה בשני הטורים הנרשמים בדומה זה בשער המוסיף חלקים והם שני הטורים אשר הוכר בם השתוף מהבלתי שתוף |
|
עוד ינשא על כל מדרגה מן המספרים הגדולים מה שבדומה לה מן המדרגות המספרים הקטנים מוכה במנין הגעות החלקים אלא אחד |
|
וכאשר עשית זה בכל מדרגות הטור הגדול הקשת בין כל מדרגה ממנו ודומה לה ממדרגות הטור הקטן ר"ל בעל המספרים הקטנים שאתה תמצא כל זה ביחס הכפל המוסיף חלקים הגעתם הוא מנין הכפלים ר"ל שמנין החלקים לא יומרו |
|
ואמנם החלק השני מזה המין והוא אשר יתרבו בו הכפלים ומנין החלקים והגעתם יחד |
|
הנה מלאכת הולדתם בהנחת המספרים הטבעיים אשר התחלתם ג' בטור |
|
עוד תניח תחת ג' ח' ותשלים הטור השני אשר התחלתו ח' בתוספת הנפרדים הטבעיים אשר התחלתם ז' |
|
ותוסיף ז' על ח' ותעמיד מה שהתקבץ במדרגה השנית וזה ט"ו |
|
עוד תוסיף על ט"ו ט' ותעמיד מה שהתקבץ במדרגה |
|
וכן תוסיף על השלישית י"א ותעמיד מה שהתקבץ ברביעית |
|
ותעשה כמו זה בשאר מדרגות הטור השני עד שתגיע לנכח סוף מה שהנחת מן המספרים הטבעיים בטור הראשון |
|
עוד נקיש כל מדרגה בדומה לה כמו שתראה בשני אלו הטורים |
| טו | יד | יג | יב | יא | י | ט | ח | ז | ו | ה | ד | ג |
| רכד | קצה | קסח | קמג | קכ | צט | פ | סג | מח | לה | כד | טו | ח |
| 15 | 14 | 13 | 12 | 11 | 10 | 9 | 8 | 7 | 6 | 5 | 4 | 3 |
| 224 | 195 | 168 | 143 | 120 | 99 | 80 | 63 | 48 | 35 | 24 | 15 | 8 |
| The species of the multiple superpartient ratio, of which the multiples, the number of the parts and their values all increase naturally, are revealed with God's help. | ויראו לך בע"ה מיני הכפל המוסיף חלקים אשר התרבו כפליהם ומנין חלקיהם והגעות חלקיהם כמו כן התרבות טבעי נמשך |
| Thus, the five ratios and the ten numeral relations were discussed and stated in a remarkable discussion, as needed, without multiplicity and lengthiness. | הנה כבר דברנו על היחסים החמש וההקשות המספריות העשרה ואמרנו בם מאמר מופלג כפי הצורך מבלתי רבוי ואריכות |
The Discussion on the Technique of Producing the Ratios from Equality |
המאמר על התחבולה בהולדת היחסים מן השווי | ||||||||||||||||||||||||||||||||
| Following what was said we present the way to produce these five ratios from equality by a basic valid coherent technique, which follows natural matters in its generality and truth of interpretation. | וכבר ימשך למה שקדם מדברינו שנביא הצד בהולדת אלו היחסים החמש מן השווי בתחבולה שרשית קיימת בלתי מבולבלת תדמה הענינים הטבעיים בכללותה ואמתת פירושה | ||||||||||||||||||||||||||||||||
| The fruit of what will be noted of this is very precious and plentiful. | והפרי במה שנזכור מזה יקר ונכבד מאד | ||||||||||||||||||||||||||||||||
| All the more so for whoever examines my investigation of the metaphor of the knowledge of good. | וכל שכן אצל מי שבחן מה שאעיין בו מדמיון הידיעה לטוב | ||||||||||||||||||||||||||||||||
| For the good is determinate in the soul, its likeness and relation are known, and the souls are drawn to it. | כי הטוב אצל הנפש מוגבל וידוע המשלו וחברו ונמשכו לו הנפשות | ||||||||||||||||||||||||||||||||
| Know, that it is first for all that is uncompounded by itself, preserving its perception in all that its nature generates, and that the evil, in its disgrace, is infinite, indeterminate, does not branch out from a root, and does not follow an interpretation, indeed, it is a change of the nature, which is good. | ותדע שהוא ראשון לכל מה שיתפרד מעצמו מתמיד ההרגש בכל מה שיתילד מטבעו ושהרע בגנותו בלתי בעל תכלית ולא מגיע אל גבול ולא יסתעף משרש ולא נמשך על פירוש אמנם הוא שנוי הטבע אשר הוא טוב | ||||||||||||||||||||||||||||||||
| Their deviation from their primary nature is a changing deviation, indeterminate, and without order. | הנה היציאה מטבעם הראשון להם יציאה מתחלפת לא תכלל בגבול ולא תתקיים בסדר | ||||||||||||||||||||||||||||||||
| Therefore, a rational capacity does not hold them by a rational hold, but recognizes them when they deviate from nature. | ולזה לא יאחזהו כח מדבר אחיזה מושכלת אבל יכירהו בהתחלפו לטבע | ||||||||||||||||||||||||||||||||
| For example: the rust in the transparent mirror and the injustice of the right soul that are incomprehensible by way of definition and determination, since they have no essence, but are known by the change of the substance in which they were set and embedded. | דרך משל אומר כחלודה במראה הספירית והעול בנפש הישרה שהם בלתי מושגים בדרך הגדר וההגבלה אחר שאין עצמיות להם ואמנם יודעו בהשתנות העצם אשר נקבעו ונטבעו בו | ||||||||||||||||||||||||||||||||
| Yet, if we find determined ways for these five ratios, by which they are produced from equality and, inversely, reduced to equality, whereas equality is undoubtedly good and virtue, then the ratios also share the same virtue and joined to the same good. | אבל אם אנחנו מצאנו לאלו היחסים החמש דרכים מוגבלים בהם יתילדו מהשווי ויותכו בהפוכם אל השווי והיה השווי בלי ספק טוב וחשיבות היו היחסים גם כן משותפים לאותו החשיבות ונקשרים באותו הטוב | ||||||||||||||||||||||||||||||||
| Since their production from it is ordered, follows a determination, and preserves the capacity of their root, from which they are produced, as the seed preserves the capacity of the tree, since the seed becomes a tree also. | אחר שהיה התילדם ממנו מסודר נמשך על גבול שומר לכח שרשם אשר ממנו יתילדו כמו שישמור הגרגיר כח האילן אחר שכבר ישוב גם כן הגרגיר אילן | ||||||||||||||||||||||||||||||||
| So these ratios are produced from equality and return to equality, however this is not by accident, rather a technique and a correlation are needed, as the cultivation of land is needed for the seed and the tree to sprout. | וכן אלו היחסים יתילדו מן השווי וישובו כמו כן אל השווי אבל לא יהיה זה בהזדמן אבל כבר נצטרך בו אל מלאכה וחבור כמו שנצטרך בענין הזרע והאילן על עבודת הקרקע קודם שיצא | ||||||||||||||||||||||||||||||||
| For it is impossible to reach the end of an assumption, be it a theoretical assumption or a practical assumption, except by using the assistance of external things, which have some similarity to that assumption, and are related in some determinate order, through the guidance of which we reach the assumption. | כי אי אפשר להגיע אל תכלית דרוש מן הדרושים דרוש מדעי היה או דרוש מעשי אם לא בהעזר מדברים מחוץ יהיה להם קצת התדמות לאותו הדרוש ויחוברו על סדר מה מוגבל נגיע עם ההדרכה עליהם אל הדרוש | ||||||||||||||||||||||||||||||||
| Because of this, Euclid stated the relation as one of the things from which knowledge exists and by which practice is reached. | ולזה שם אקלידס החבור אחד מהדברים אשר מהם תהיה הידיעה ובהם נגיע אל המעשה | ||||||||||||||||||||||||||||||||
| Therefore, in the production of each of these ratios, we also place three numbers in three terms, in order to reach the relation through them. | ולזה גם כן נניח אנחנו בהולדת כל אחד מאלו היחסים שלשה מספרים בשלשה מדרגות להגיע בם אל ההתיחסות | ||||||||||||||||||||||||||||||||
| For the least relation possible is in three determinations. | כי ההתיחס בפחות מה שיהיה הוא בשלשה גבולים | ||||||||||||||||||||||||||||||||
| In relation, one is assisted by things that are apart from that which has the similarity of the ratio. | ואמנם יעזרו בהתיחסות מהדברים אשר מחוץ למה שבו מהתדמות היחס | ||||||||||||||||||||||||||||||||
| Since it was already said that the things that assist us are apart from the investigation of the assumption, they should be similar to the assumption. | ולפי שאנו כבר אמרנו שהדברים אשר נעזר בם מחוץ אל החקירה מהדרוש ראוי שיהיו מתדמים לדרוש | ||||||||||||||||||||||||||||||||
| These three terms are called first, second and third. | ויקראו אלו שלשת המדרגות ראשונה ושניה ושלישית | ||||||||||||||||||||||||||||||||
| Each term among them is limited by a determination which it does not surpass: | ותדבק כל מדרגה מהן בגבול לא תעברהו | ||||||||||||||||||||||||||||||||
|
אם הגבול במדרגה הראשונה הוא שנניח תחתיה לעולם כמו המספר אשר בה | ||||||||||||||||||||||||||||||||
|
ואמנם הגבול במדרגה השנית הוא שנניח תחתיה לעולם כמו המספר אשר בה וכמו המספר אשר במדרגה הראשונה | ||||||||||||||||||||||||||||||||
|
ואמנם הגבול אשר במדרגה השלישית הוא שנניח תחתיה כמו המספר אשר בה וכמו המספר אשר במדרגה הראשונה וכפל אשר במדרגה השנית | ||||||||||||||||||||||||||||||||
| This is the general technique by which each of these ratios is produced. | זה הוא מה שהוא כולל במלאכה אשר בה יתילד כל אחד מאלו היחסים | ||||||||||||||||||||||||||||||||
| Yet, what is particular in it for each of these ratios is: | ואמנם מה שהוא מיוחד בה לכל אחד מאלו היחסים | ||||||||||||||||||||||||||||||||
|
כי אשר תיחד המלאכה בהולדת יחס הכפל השווי שיהיו המספרים הראשונים המונחים במדרגות הג' אשר מהם יתילד זה היחס מספרים שוים שומרים השווי בהצטרפם | ||||||||||||||||||||||||||||||||
|
וכשנשמור בם התנאי הכולל הנזכר יראו אלינו מספרים ביחס הכפל השניי והיה המין הראשון ממיני זה זה היחס | ||||||||||||||||||||||||||||||||
|
ואם שמנו זה המין הנראה מונח והוספנו בו התנאי הכולל הנזכר ר"ל העשות הגבולים השלשה במדרגות השלשה יראה לנו המין השני ממיני הכפל והוא הכפל השלישיי | ||||||||||||||||||||||||||||||||
|
ואם שמנוהו גם כן מונח והשבנו בו במעשה הראשון יראה לנו המין השלישי והוא הכפל הרביעיי | ||||||||||||||||||||||||||||||||
|
וכפי זה הדרך לא נסור מזה המעשה יראו לנו מיני הכפל ראשון ראשון כפי המשכם בטבע | ||||||||||||||||||||||||||||||||
|
ובחן זה בשני טורים אלו | ||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||
|
וקחם לשרש למה שימשך להם | ||||||||||||||||||||||||||||||||
|
ואמנם אשר הוא מיוחד בזאת המלאכה להוליד יחס המוסיף חלק הנה הוא שיהיו המספרים המונחים אשר מהם תרצה להוליד המוסיף חלק מתיחסים ביחס הכפל ויהיה הגדול מהם במקום הראשון והקטן מהם במקום השלישי עוד תעשה בם הסבוב הכולל אשר זכרנו | ||||||||||||||||||||||||||||||||
|
כי ימשך לזה שיראו אלינו מיני המוסיף חלק כפי המשכם בטבע | ||||||||||||||||||||||||||||||||
|
ר"ל שהמספרים המונחים אם היו עליהם תחלת מיני הכפל ר"ל השניי יראו מהם מיני המוסיף חלק ר"ל המוסיף חצי | ||||||||||||||||||||||||||||||||
|
ואם היו על היחס השני ממיני הכפל ר"ל השלישיי יראה לנו גם כן השני מהמוסיפי חלק ר"ל המוסיף שליש | ||||||||||||||||||||||||||||||||
|
וכמו כן מה שאחר זה | ||||||||||||||||||||||||||||||||
| Examine it from this diagram: | ותבחנהו מזאת הצורה | ||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||
|
ואשר ייחד תולדת המוסיף חלקים הוא שיהיו המספרים המונחים מתיחסי' ביחס המוסיף חלק ויהיה הגדול מהם במקום הראשון והקטן מהם במקום השלישי עוד נעשה בם הסבוב הכולל הנזכר | ||||||||||||||||||||||||||||||||
|
כי הוא יראה לנו המספרים על יחס מין מהמוסיף חלקים מסודרים בקירוב מהטבע בסדור המוסיף חלק המונח וזה יהיה כן | ||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||
|
ואשר ייחד הולדת יחס הכפל המוסיף חלק הוא שיהיו המספרים המונחים מתיחסים ביחס המוסיף חלק גם כן אלא שהגדול מהם יהיה במקום השלישי והקטן מהם במקום הראשון | ||||||||||||||||||||||||||||||||
|
ויראה לנו הסבוב הכולל המותנה מין מהכפל המוסיף חלק הוא בקרוב מהטבע ר"ל מהתחלת מיני הכפל המוסיף חלק בכמו מדרגת המין המוסיף חלק המונח ויהי כן | ||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||
|
ואשר ייחד הולדת יחס הכפל המוסיף חלקים הוא שיהיו המספרים המונחים מתיחסים יחס המוסיף חלקים ויהיה הקטן מהם במקום הראשון והגדול מהם במקום השלישי | ||||||||||||||||||||||||||||||||
|
וכאשר נסובב בהם המעשה אשר הוא כולל לכל היחסים יראה לנו מין מהכפלים המוסיף חלקים מסודרים בסוגו כסדר המוסיף חלקים המונח כסוגו וזה כמו שתראה הנה | ||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||
| It has already become clear that all these ratios that were brought here as an example were produced from the line of the three equal terms, which is the line of the units written in red. | הנה כבר התבאר שכל אלו היחסים שהמשלנו אותם בכאן אמנם יתילדו מהטור השוה המדרגות השלשה והוא טור האחדים הנכתבים באודם | ||||||||||||||||||||||||||||||||
| If non-units were placed in this line, as long as they are equal numbers, if we operated on them in the ways we described, then all that we saw of the matters required in the production of these ratios twill necessary follow, except that what will be seen from this is not its cause; rathert its cause is the equality of the units. | ואלו הנחנו כמו כן באותו הטור בלתי האחדים רק שיהיו מספרים שוים ועשינו בם מה שעשינו אותו בדרכים אשר תארנו יחוייב שימשך לזה כל מה שנראה לנו מהענינים המתחייבים בהולדת אלו היחסים אלא שאשר נראה מזה אינו עלתו אבל עלתו שווי האחדים | ||||||||||||||||||||||||||||||||
| It can be known that the species of the superpartient ratio produced by this technique are indeed the first of these ratios. | וכבר יתכן לנו שנדע כי מיני המוסיף חלקים אשר יתילדו בזאת המלאכה אמנם הם המינים הראשונים מאלו היחסים | ||||||||||||||||||||||||||||||||
| By "the first species" I mean those whose values of their parts exceed the number of their parts by one, as the superbitertian ratio, the supertriquartan ratio, the superquadriquintan ratio, the superquintisextan ratio, and what follows likewise. | ורצוני במינים הראשונים מאלו מהם אשר יהיו הגעות חלקיהם יותר מוסיפים ממנין חלקיהם באחד כמו המוסיף שני שלישים והמוסיף שלשה רביעיות והמוסיף ארבעה חומשים והמוסיף חמשה שתויות ומה שיעבור זה ממה שידמהו | ||||||||||||||||||||||||||||||||
| The rest of the species of the superpartient ratio, such as the superbiquintan ratio, the supertriquintan ratio, or the supertriseptan ratio, or likewise - the technique for their production is as we stated about in the measure of superpartient ratio. | ואמנם שאר מיני המוסיף חלקים כמו המוסיף שני חומשים והמוסיף שלשה חומשים או המוסיף שלשה שבעיות או מה שהוא דומה זה הנה המלאכה בהולדתם אמנם היא כמו מה שהגדנו עליה בשעור המוסיף חלקים | ||||||||||||||||||||||||||||||||
| When we want the species of the multiple superpartient ratio in this technique, it is necessary that the given species of the superpartient ratio that will produce it are the first species mentioned, whose value of their parts exceeds the number of their parts by one. | וכן גם כן ראוי לנו כשנרצה מיני הכפל המוסיף חלקים בזאת המלאכה שיהיו המינים המונחים מהמוסיף חלקים להוליד זה הם המינים הראשונים אשר זכרנו שהגעת חלקיהם תוסיף על מנין חלקיהם אחד | ||||||||||||||||||||||||||||||||
| This alone is a sufficient discussion of the instruction of whichever species of the multiple superparticular ratio and whichever species of the multiple superpartient ratio that are produced in the mentioned explanation; the rest of the kinds of these ratios are more distant from the way of their kinds, and because of this they are perhaps not visible except by a more complex technique than the one that we have just completed recording. | וזה בלבד מאמר מספיק בהוראה על אי זה מיני הכפל המוסיף חלק ואי זה מיני הכפל המוסיף חלקים יתילדו בבאור הנזכר וששאר סוגי אלו היחסים יתרחקו מדרך סוגיהם ולזה אולי לא יראו אלא במלאכה היא יותר רבת ההרכבה מזאת אשר השלמנו לזכרה עתה | ||||||||||||||||||||||||||||||||
| God is the Knower. | והאל היודע | ||||||||||||||||||||||||||||||||
| This is for you the conception of the categorization of the relative quantity | וזה לך ציור חלוקת הכמה המצטרף | ||||||||||||||||||||||||||||||||
| This, may God lead you straight, is enough for the end of the first section of the book of arithmetic as described by the Pythagorean Nicomachus al-Gehrasīnī. | הנה זה יישירך השם מספיק בסוף המאמר הראשון מספר הארתמאיטיקא כפי מה שתיארו ניקמאכוש אלגהרשיני הפתאגורי | ||||||||||||||||||||||||||||||||
| Revised in al-Andalus by Abū Sulaymān Rabīʽ ben Yaḥyā usquf Elvira. | ותקנו באנדלס אבו סלימאן רביע בן יחיי אסקף אלבירה | ||||||||||||||||||||||||||||||||
| Find help in its investigation and study | והעזר בעיון וההגיה בו | ||||||||||||||||||||||||||||||||
| May God in His mercy lead you straight to understand and find your wishes that are useful for your end, Amen. | והאל ברחמיו יישירך להבין ולמצוא חפציך בו המועילים לאחריתך אמן | ||||||||||||||||||||||||||||||||
| The first section of the book of arithmetic is complete. | נשלם המאמר הראשון מספר הארתמאטיקא | ||||||||||||||||||||||||||||||||
| Praise be to God. | והתהלה לאל | ||||||||||||||||||||||||||||||||
| The second section follows it. | יבא אחריו המאמר השני בג"ה |
Book Two |
המאמר השני מספר הארתמאטיקא | ||||||||||||||||||||||||||||||||||||
The First Section: Reducing the Ratios to Equality |
המאמר הראשון ממנו בהתכת היחסים אל השווי | ||||||||||||||||||||||||||||||||||||
| He [= Nicomachus] said: the principle and the foundation is the thing from which another thing is composed and into which another thing is decomposed. | אמר שהפנה והיסוד הוא הדבר אשר הורכב ממנו דבר אחר ויותך אליו דבר אחר | ||||||||||||||||||||||||||||||||||||
For example:
|
כמו אותיות האלפא ביתא כי יאמר בם שהן פנות לכתבים המונחים המחוברים מהם הנתכים אליהם | ||||||||||||||||||||||||||||||||||||
|
וכן הנעימות הן פנות לכל הלחנים אחר שהיו הלחנים מחוברים מהם נתכים אליהם | ||||||||||||||||||||||||||||||||||||
|
וכן גם כן אלו היסודות הארבעה ר"ל האש והאויר והמים והארץ יאמר שהם פנות ופשוטים לכל הנופלים תחת ההויה וההפסד אחר שחבורם מהם והתכתם אליהם | ||||||||||||||||||||||||||||||||||||
| These propositions were brought forward to demonstrate that equality is a principle of the relative quantity, from which it yields and to which it is reduced. | ואמנם הקדמנו אלו ההקדמות להראות שהשווי פנה לכמות המצטרף ושהוא ממנו צמח ואליו יותך | ||||||||||||||||||||||||||||||||||||
| As for the absolute quantity, its first principle, from which it is produced, and which can be added endlessly, is the one. | ואמנם הכמה הנפרד הנה פנתו הראשונה ובו התילד ואיפשר להוסיף בו אל מה שאין תכלית לו הוא אחד | ||||||||||||||||||||||||||||||||||||
| What is produced from equality, and reduced to it, is common to all the relations. | ואמנם מה שיתילד מן השווי ויותך אליו הנה הוא כולל לכל הצרופים | ||||||||||||||||||||||||||||||||||||
| A proof will be brought, by demonstration and true meaning, that equality is a cause of the relative quantity, from which it is produced, by which it yields when added, and into which it is consumed when subtracted and divided. | ונביא ראיה במופת ואמתות שהשווי סבה לכמות המצטרף אשר ממנו היה הולדתו ובו צמח בהתוספו ואליו יכלה כאשר חוסר ונתך | ||||||||||||||||||||||||||||||||||||
From the general technique one knows that when setting three numbers, such that the ratio of the first of them to the second is as the ratio of the second to the third [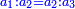 ], in any species of the five ratios mentioned in the first section, these three given numbers are also such that the ratio of the third to the second is also as the ratio of the second to the first [ ], in any species of the five ratios mentioned in the first section, these three given numbers are also such that the ratio of the third to the second is also as the ratio of the second to the first [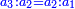 ], when the first is the smaller, the second is the mean, and the third is the greater. ], when the first is the smaller, the second is the mean, and the third is the greater.
|
ומהתחבולה הכוללת לזה הוא שתדע שכאשר תניח שלשה מספרים יחס הראשון מהם אל השני כיחס השני אל השלישי באי זה מהמינים היה מהיחסים החמש אשר זכרנום במאמר הראשון הנה אלו מספרים שלשה אם הונחו כן היה יחס גם כן השלישי אל השני כיחס השני אל הראשון והראשון הוא הקטן והשני הוא האמצעי והשלישי הוא הגדול | ||||||||||||||||||||||||||||||||||||
|
ואם רצה רוצה להשיבם אל השווי הנה ישליך מהאמצעי כמו הקטן עוד ישליך מהגדול כמו מה שישאר מהאמצעי וכמו מהאמצעי | ||||||||||||||||||||||||||||||||||||
|
ואם השתוו המספרים השלשה הנה כבר היה מה שרצינו | ||||||||||||||||||||||||||||||||||||
|
ואם לא הנה כבר יצאו ממין צרופם ושבו אל מין אחר הוא יותר קודם בטבע ממין היחס אשר היו ממנו ראשונה | ||||||||||||||||||||||||||||||||||||
|
עוד תעשה בו כמו המעשה הראשון | ||||||||||||||||||||||||||||||||||||
|
ואם השתוו הנה מצאנו מבוקשנו | ||||||||||||||||||||||||||||||||||||
|
ואם לא הנה כבר יצאו מאותו המין גם כן אל מין יותר רחוק מן הראשון בטבע | ||||||||||||||||||||||||||||||||||||
|
ולא תסור מעשות כן תמיד עד שישתוו | ||||||||||||||||||||||||||||||||||||
| Example: | ומשל זה | ||||||||||||||||||||||||||||||||||||
|
נניח השווי בטור בעל שלשה מדרגות | ||||||||||||||||||||||||||||||||||||
|
ונסדר אליו טור שני מתיחס ביחס הכפל | ||||||||||||||||||||||||||||||||||||
|
עוד נסדר בם טור שלישי על יחס המוסיף חלק | ||||||||||||||||||||||||||||||||||||
|
ונמשיך בזה טור רביעי על יחס המוסיף חלקים | ||||||||||||||||||||||||||||||||||||
|
עוד נניח בטור החמשי מספרים מתיחסים ביחס הכפל המוסיף חלק | ||||||||||||||||||||||||||||||||||||
|
ונחתום הצורה בטור ששי מתיחס המדרגות ביחס הכפל המוסיף חלקים | ||||||||||||||||||||||||||||||||||||
| As in this diagram: | כפי מה שבצורה | ||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||
|
הנה זה הטור שנכוין אליו מאלו הטורים ועשינו בו מלאכת ההתכה אשר זכרנוה נשיבהו בזה אל יחס טור הוא יותר קרוב ממנו אל השווי ר"ל שהוא יותר קרוב אל טור הגבולים השוים | ||||||||||||||||||||||||||||||||||||
|
עוד לא נסור מעשות זה בו עד שישובו מדרגותיו אל יחס טור השווי עצמו | ||||||||||||||||||||||||||||||||||||
| What was said is enough, as opposed to extending examples and the long repetition that are not adequate for the one who knows that the fulfillment of the arts is not by multitude of examples, but by setting determinations/definitions. | ויספיק לך מה שאמרנו מלהרבות ההמשלים וההכפלה הארוכה אשר לא יאותו למי שידע שהשלמת המלאכות לא תהיה ברבוי ההמשלים אבל בתקון הגבולים | ||||||||||||||||||||||||||||||||||||
| It is now clear that the procedure, which was brought forward in the first section for the production of the ratios, reverses itself when trying to reduce the ratios to equality. | וכבר התבאר כי המעשה אשר הקדמנוהו להולדת היחסים במאמר הראשון הוא בעצמו יתהפך הנה כאשר נשתדל להתיך אותם היחסים אל השווי |
The Discussion on the Arrangement of the Terms of each Ratio of two Given Numbers from Superparticulars |
הדבור בסדור גבולי כל יחס מונח שני המספרים מיחסי המוסיף חלק | ||||||||||||||||||||||||||||||||||||||||||
| Since we very much need to set successive proportional numbers of the sesquialter ratio, the sesquitertian ratio, the sesquiquartan ratio, or other ratios of the superparticular ratios, we should bring an artful extraction procedure for it and a technique that will verify its assumption, in order that the investigation of what we investigate will be without confusion. | וראוי אחר שכבר נצטרך הרבה אל שנניח מספרים נמשכים מתיחסים מהמוסיף חצי או המוסיף שליש או המוסיף רביע או זולת מה מהמוסיף חלק אחר שנביא הוצאה מלאכותית לזה ותחבולה תאמת לנו הסברא בה כדי שתהיה דרישתנו למה שנדרשנו מזה מבלתי בלבול | ||||||||||||||||||||||||||||||||||||||||||
|
ונאמר שיחס המוסיף חצי הוא ראש יחסי המוסיף חלק כמו שנגלה במה שקדם | ||||||||||||||||||||||||||||||||||||||||||
|
ושתחלת שני מספרים פועלים יחס המוסיף חצי הם שנים ושלשה | ||||||||||||||||||||||||||||||||||||||||||
|
וכאשר נרצה לבאר איך נוציא הגבולים הנלוים בזה היחס | ||||||||||||||||||||||||||||||||||||||||||
|
נסדר מדרגות הכפל השניי בטור והם מדרגות זוג הזוג | ||||||||||||||||||||||||||||||||||||||||||
|
ונשליך המדרגה הראשונה לפי שהיא האחד ואין חצי לו | ||||||||||||||||||||||||||||||||||||||||||
|
עוד נניח תחת כל מדרגה המספר הפועל עמה יחס הדמיון וחצי ר"ל יחס המוסיף חצי ויתחדש טור שני חסר מהטור הראשון מדרגה והיא אשר השלכנו בנוכח האחד | ||||||||||||||||||||||||||||||||||||||||||
|
עוד נשוב ונשליך כמו כן המדרגה הראשונה מזה הטור השני לפי שהוא שלשה ואין חצי לו | ||||||||||||||||||||||||||||||||||||||||||
|
ונניח תחת כל אחת ממדרגותיו הנשארות המספר הפועל עמו יחס המוסיף חצי ויתחדש טור שלישי חוסר מהטור השני מדרגה והיא המושלכת נגד השלשה | ||||||||||||||||||||||||||||||||||||||||||
|
עוד נשוב גם כן ונשליך המדרגה הראשונה מזה הטור השלישי לפי שהוא תשעה ואין לו חצי | ||||||||||||||||||||||||||||||||||||||||||
|
ונניח תחת כל אחת ממדרגותיו המספר הפועל עמו יחס המוסיף חצי הנה יתחדש טור רביעי חוסר מהטור השלישי מדרגה והיא אשר השלכנו כנגד התשעה | ||||||||||||||||||||||||||||||||||||||||||
|
וכמו כן נחדש טור חמישי וששי ולמעלה מזה כמו שתראה בזאת הצורה | ||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||
|
וכבר התבאר שתחלת מה שימצא יחס המוסיף חצי בשני גבולים | ||||||||||||||||||||||||||||||||||||||||||
|
עוד התבאר בזאת הצורה איך נמצאהו בשלשה גבולים עוד בארבעה עוד בחמשה עוד בששה | ||||||||||||||||||||||||||||||||||||||||||
| Through the technique, by which this diagram is made, terms are added in this ratio endlessly, and no term is missing in any available space in which this technique is not evident. | והמלאכה שנעשית בה זאת הצורה כבר יתוספו בה הגבולים בזה היחס אל מה שאין תכלית לו ולא יבצר מהם גבול בשום פנוי שלא תראה בו התחבולה | ||||||||||||||||||||||||||||||||||||||||||
|
ואם רצינו להוליד מספרים כפי זה המשל ביחס המוסיף חלק השלישי | ||||||||||||||||||||||||||||||||||||||||||
|
נסדר מה שבטור הכפל השלישי הנמשך על יחס דמיון א' ג' ט' כ"ז פ"א רמ"ג | ||||||||||||||||||||||||||||||||||||||||||
|
ונסדר טורים תחת זה הטור נעמיד תחת כל מספר כמהו וכמו שלישיתו מה שאפשר בו זה | ||||||||||||||||||||||||||||||||||||||||||
|
ונצייר צורתו כמו מה שציירנו הראשונה אשר לפניה ויראו בה מדרגות הגבולים ביחס המוסיף שליש מבלתי שיחטא בגבול מהם כמו שתראה בזאת הצורה אשר לפניך | ||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||
|
וכמו כן כאשר נרצה ביחס המוסיף חלק הרביעיי הנמשך על יחס דמיון א' ד' י"ו ס"ד | ||||||||||||||||||||||||||||||||||||||||||
|
וכמו כן מה שילוה לזה מין ממיני המוסיף חלק | ||||||||||||||||||||||||||||||||||||||||||
| The basis of the technique of this diagram is to place the successive terms of the first line in the multiple ratio, after which the superparticular ratio of the required terms is nameed, i.e. the multiple that yields the denominator of the part. | ואמנם היסוד במלאכת זאת הצורה שנשים מדרגות הטור הראשון נמשכים על יחס הכפל אשר הוא נקרא ליחס הדרוש הגבולים מיחסי המוסיף חלק ר"ל הכפל אשר ממנו מעמד אותו החלק | ||||||||||||||||||||||||||||||||||||||||||
| When it is produced in this diagram by the mentioned technique, it is evident and clear that the numbers that are placed in the angles [the main diagonal] of each diagram are the numbers that are in the first given line of the following diagram. | וכאשר עשית אותו מזאת הצורה במלאכת הנזכרת הוא גלוי מבואר שהמספרים אשר יפלו בזויות מכל צורה הם המספרים אשר בטור הראשון המונח בצורה אשר תמשך לה | ||||||||||||||||||||||||||||||||||||||||||
| Here are other notable and wonderful properties that you will understand through your good examination by the will of God. | ובכאן עניינים אחרים נכבדים ונפלאים תעמוד עליהם עם טוב בחינתך בהם ברצון השם | ||||||||||||||||||||||||||||||||||||||||||
| Revealing which ratio of the superparticular ratios is found in a composition, i.e. from the composition of which of the ratios follows whichever multiple ratio required. | על גלוי אי זה יחס מיחסי המוסיף חלק הנמצא בחבור ר"ל נמצא בחבוריהם יחס מונח ופי' איזה יחסים הם אשר יתחייב מחבורם יחס איזה כפל שתדרוש בחבור יחס מונח מיחסי בעל הכפלים | ||||||||||||||||||||||||||||||||||||||||||
|
אמנם המין הראשון מבעל הכפלים והוא השניי הנה הוא יתחבר ויתילד באמצעות שני המינים הראשונים ממיני המוסיף חלק והם המוסיף חצי והמוסיף שליש | ||||||||||||||||||||||||||||||||||||||||||
|
משל זה שהארבעה כמו השלשה וכמו שלישיתם | ||||||||||||||||||||||||||||||||||||||||||
|
|
והשלשה כמו השנים וכמו חציו | ||||||||||||||||||||||||||||||||||||||||||
|
והנה הארבעה אצל השנים ביחס הכפל השניי כמו שתראה בזאת הצורה | ||||||||||||||||||||||||||||||||||||||||||
|
ובהפך זה המאמר יתבאר שהכפל השניי יותך אל המוסיף חצי והמוסיף שליש | ||||||||||||||||||||||||||||||||||||||||||
|
משל זה שאם לקחנו שני מספרים ביחס הכפל השניי כמו הששה והשלשה שאנו נמצא ביניהם אמצעי מן המספר יותך אצלו יחס שני הקצוות המונחים מהכפל השניי על שני היחסים הנזכרים מיחסי המוסיף ואם כן יותך אליהם חלק | ||||||||||||||||||||||||||||||||||||||||||
|
והאמצעי בין הששה והשלשה הם ארבעה | ||||||||||||||||||||||||||||||||||||||||||
|
הנה הששה כאשר הוקשו אל הארבעה היה המוסיף חצי | ||||||||||||||||||||||||||||||||||||||||||
|
והארבעה אצל השלשה תוסיף שליש | ||||||||||||||||||||||||||||||||||||||||||
|
וכאשר יותך אמנם יותך אליהם | ||||||||||||||||||||||||||||||||||||||||||
|
ואמנם המין השני מבעל הכפלים ר"ל הכפל השלישיי אמנם יולד מהמין הראשון המוסיף חלק ר"ל החצי והמין הראשון מבעל הכפלים ר"ל השניי | ||||||||||||||||||||||||||||||||||||||||||
|
משל זה שי"ח כמו י"ב וכמו חציו | ||||||||||||||||||||||||||||||||||||||||||
|
|
וי"ב כפל ו' | ||||||||||||||||||||||||||||||||||||||||||
|
אבל י"ח אצל ו' כיחס הכפל השלישיי | ||||||||||||||||||||||||||||||||||||||||||
|
וכמו כן גם כן תמצאהו אלא שלא יחוייב שנשים האמצעי י"ב אשר הוא כפל ו' אבל נשים במקומו ט' אשר הוא כמו ו' וכמו חציו | ||||||||||||||||||||||||||||||||||||||||||
|
וכבר המשלתי לך שתי הצורות | ||||||||||||||||||||||||||||||||||||||||||
|
ובהפך זה המאמר יתבאר שהכפל השלישי כאשר יותך אמנם יותך אל הכפל השניי והמוסיף חצי | ||||||||||||||||||||||||||||||||||||||||||
|
ובחן זה באי זה משני מספרים תרצה אם תניחם ביחס הכפל השלישיי ותמצאהו כן | ||||||||||||||||||||||||||||||||||||||||||
|
ואמנם אם יחובר הכפל השלישיי והמין השני מהמוסיף חלק יתילד מזה הכפל הרביעיי | ||||||||||||||||||||||||||||||||||||||||||
|
וכאשר יותך הכפל הרביעיי הנה הוא יותך אל הכפל השלישיי ואל המוסיף חלק השניי | ||||||||||||||||||||||||||||||||||||||||||
| The rule of the discussion is that every species of the multiple ratios, when it is composed with its corresponding species of the superparticular ratios, the closest type that follows it among the multiple ratios is composed from them. | וכלל המאמר שכל מין ממיני הכפל כאשר הורכב עם דומה לו במדרגה ממיני המוסיף חלק הנה יתחבר מהם המין אשר ילוה אליו בקרוב שבמדרגות אליהם ממיני הכפל | ||||||||||||||||||||||||||||||||||||||||||
| This is the full discussion on the relative quantity with all the aspects of its relations, while keeping as much as possible the completion of the properties by a brief discussion about that which the scholars cannot do without. | זהו כלל המאמר בכמה המצטרף על כל צדי צירופיו עם תכלית מה שאיפשר מהשמירה בהשלמת הענינים עם קצור המאמר במה שאי אפשר למתלמדים בלעדיו |
[Absolute Quantity] |
|
The Discussion of the Quality of the Absolute Numbers that are Potentially Commensurable with the Geometric Shapes |
הדבור בתואר המספרים הנפרדים המשותפים בכחם לתמונות המידותיות |
| The beginning of this discussion on the geometrical shapes: | ותחלת זה הדבור בתמוניות |
| Now we note what remains of the things that belong to the absolute quantity, which requires a more intensive investigation than all that we discussed in the first section. | אמנם עתה הנה אנחנו זוכרים מה שנשאר מהדברים המתחייבים לכמה הנפרד ממה שיצטרך לחקירה יותר חזקה מכל מה שדברנו עליו במאמר הראשון |
| Indeed, when we wish to note it, there is something that we need in order to derive evidence concerning another. | כי כאשר אנחנו רוצים לזכרו הנה הוא דבר נצטרך אליו לקחת ממנו ראיה על זולתו |
| The wise men used to praise the explanation of some sciences through others. | והחכמים היו משבחים מאד לפרש קצת החכמות בקצת |
| Of the things whose explanation should be prefaced, it should be said that: | ומהדברים אשר ראוי להקדים בבאורם שנאמר כי |
|
מהמספרים מה שהוא דומה לקוים בסדרם |
|
ומהם מה שהוא דומה לשטח |
|
ומהם מה שהוא דומה למעוקב |
|
ומהם מה שהוא דומה לכדור |
|
ומהם מה שהוא דומה ללבנה בחסרון הגובה מהאורך והרחב |
|
ומהם מה שהוא דומה לעמוד בחסרון האורך והרחב מהגובה |
|
ומהם מה שהוא דומה לקשת |
| Likewise for each of the things of geometry, i.e. of measure - these shapes have a likeness in numbers. | וכמו כן לכל אחד מהדברים אשר להנדסה ר"ל המדות יש דמיון לאותם התמונות במספרים |
| For geometry is based on quality more than on quantity. | כי ההנדסה לאיכות יותר דבקה מאשר לכמות |
| Look carefully on what was mentioned about the precedence of the number over the measures in the introduction to the number, because it was noticed and discussed there. | ותעיין מה שזכרנו מקדימת המספר למדות בפתיחת המספר כי שם שמנו אליו לב ודברנו בו |
| Before discussing the similarity of these mentioned determinations to the numbers, we say that the meaning of the alphabet letters as designating the numbers that are marked by them, which are the shapes that are seen, which the people call decimal calculation, is not in their nature, nor in their law, but they designate them only by convention and positing. | וקודם שנדבר על דמיון אלו הגבולים אשר זכרנום מהמספרים נאמר שהוראת אותיות האלפא ביתא על מה שיורו עליו מן המספרים הנרשמים בם והם הרשמים אשר אנו רואים שיקראום האנשים חשבון הכללים אינו בטבע להם ולא בדין להם כך אבל הם מורים בהסכמה ובהנחה ממנו לא זולתו |
| Those [sign] that indicate [numbers] by nature are like those [objects] visible by the sense. | ואמנם המורים בטבעם הנה הם כמו הנראים לחוש |
|
והוא שאנו כאשר נרצה לרשום אחד נניח סימן אחד |
|
וכשנרצה לרשום שנים נניח שני סמנים |
|
וכן אם נרצה שלשה נניח שלשה סמנים |
| These will be the form of the one, the form of the two, the form of the three, and so on for what follows. | ותהיה צורת האחד וצורת השנים וצורת השלשה וכן מה שאחר זה |
| When we bring this condition, we say that the one is assumed in this craft as the beginning of number, like the rank of the point in the science of geometry, which is the beginning of the line, while the point or the one are neither a line nor a number. | וכאשר הבאנו זה התנאי נאמר שאנו נניח האחד בזאת המלאכה התחלה למספר כמדרגת הנקודה במלאכת המדות אשר היא התחלת הקו מבלתי שתהיה הנקודה או האחד קו או מספר |
| As when the point is multiplied by itself, no other quantity is generated. | הנה כמו שהנקדה כאשר נכפלה בעצמה לא יתחדש גודל אחר |
| Because, when what has no magnitude is multiplied by what has no magnitude, no magnitude is generated | שמה שאין גודל לו כאשר נכפל במה שאין גודל לו לא יתחדש גודל |
| Since no being emerges from non-being. | לפי שלא יהיה יש מלא יש |
| Likewise the one, which is not a number, when it is multiplied by itself, no number is generated, as no being is generated from the multiplication of nothing. | כמו כן האחד אשר אינו מספר כאשר נכפל בעצמו לא יתחדש מספר לפי שלא יתחדש יש מכפל אין |
| Hence, the essence of the one, as it is said, is that it is the beginning of the number, but it is not a number. | הנה אם כן אמתת האחד כמו שאמרנו שהוא תחלת המספר ואינו מספר |
Plane Numbers |
|
The Discussion of the Aspect of Similarity between the Number to the Line |
הדבור בצד התדמות המספר לקו |
| The beginning of its continuity is found in duplicity. | ואמנם תחלת המשכותו אמנם ימצא בשניות |
| Furthermore, it increases continually at the rate of the addition of the natural numbers and their production. | עוד יתרבה בהמשכות כפי שעור הוספת המספרים הטבעיים וצמיחתם |
| As the number is generated by assuming two different units such that the number arises from their difference, so the line is generated by assuming two different point, such that the line arises is from their difference. | הנה כמו שהמספר יתחדש בהנחת שני אחדים נבדלים ויהיה מהבדלם המספר כן הקו אמנם יתחדש בהנחת שתי נקודות נבדלות הנה יהיה מהבדלם הקו |
|
והקו בעל משך אחד |
|
ומה שהיה בעל שני המשכים הוא שטח |
|
ומה שהיה בעל שלשה המשכים הוא גשם |
| The three-dimensional has six directions, which are said to be necessary in every solid, i.e. up, down, right, left, forward, backward. | ולבעל השלשה המשכים מה שיתחדש השש פאות אשר יאמר שהן מתחייבות לכל גשם ר"ל מעלה ומטה וימין ושמאל ופנים ואחור |
| By these six directions the motion divides the places. | ובאלו השש פאות תחלק התנועה המקומות |
| For it holds necessarily that each dimension has two opposite directions, whether one has the two directions up and down, or another forward and backward, or another right and left. | כי בהכרח שיתחייב לכל משך שתי תכליות מקבילים אם לאחד משתי התכליות מעלה ומטה ואם לאחר פנים ואחור ואם לאחר ימין ושמאל |
|
ואולי יהיה לטוען שיאמר הנה במאמר כולל שכל בעל אורך ורוחב ועומק הוא גשם וכל גשם בעל אורך ורחב ועומק |
|
וכמו כן יאמר מה שהיה לו אורך ורחב לבד הוא שטח והשטח הוא מה שהיה לו אורך ורחב לבד |
|
והקו מה שהיה לו אורך לבד ומה שהיה לו אורך לבד הוא קו |
Hence:
|
הנה השטח חסר מהגשם במשך אחד |
|
והקו מהשטח במשך אחד |
|
והנקודה חסרה מהקו במשך אחד |
| Hence, the point has no dimension at all. | הנה אם כן הנקודה אין מרחק לה כלל |
|
ואמנם היא התחלת הקו ואיננה קו |
|
וכמו כן הקו התחלת השטח ואיננו שטח |
|
והתחלת שני המשכים ואיננו שני המשכים |
|
וכמו כן השטח התחלת הגשם ואיננו גשם |
|
והתחלת שלשה המשכים |
| Thus, the numbers are also similar to these determinations, as will be described. | הנה אכן גם כן המספרים הם דומים לאלו הגבולים כפי מה שאתאר |
| Indeed, the linear numbers are all those that are set to begin with two and increase by one according to the order of the natural succession. | וזה שהמספרים הקויים הם כל מה שתניח התחלתו מהשנים עוד יתרבו באחד על סדר משך הטבע |
The Discussion of the Triangle as the Foundation of all Rectilinear Surfaces |
הדבור באשר המשולש יסוד לכל השטחים ישרי הקוים |
| The first of the rectilinear surfaces is the triangle. | ואמנם השטחים ישרי הקוים הראשון מהם המשולש |
| It is the one that has three angles. | והוא בעל השלש זויות |
| It is as a root and a beginning of all flat surfaces, such as the square, the pentagon, the hexagon, and their similar. | והוא כשרש וכהתחלה לכל השטחים הישרים כמו המרובע והמחומש והמשושה והדומה להם |
| For all of them are decomposeable into the triangle. | כי כלם יותכו אל המשולש |
| But the triangle does not decompose into anything but its likeness. | וזה המשולש לא יותך אל בלתי תמונתו |
| This is clear, because when you draw lines from the angles of whichever regular shape you wish to its center, the shape, whichever it may be, will be divided into triangles. | וזה מבואר מאשר כשתוציא מאיזה מהתמונות הישרות הקוים שתרצה קוים ישרים מזויותיהם אל מרכזה תחלק איזו תמונה שתהיה אל המשולשים |
| you find that the triangle also divides itslef into itself, unchanged by its nature and not deviating from its genus. | ותמצא המשולש יחלק גם כן עצמו אל עצמו בלתי נבדל מטבעו ולא יצא מסוגו |
| It is already visible to the sense from this figure that the triangle, the square, the pentagon, and the rectilinear shapes that follow them, when we draw in them the described lines from their centers to their angles, all of them decompose into triangles. | וכבר תראה זה בזאת הצורה לחוש שהמשולש והמרובע והמחומש ומה שאחריהם מהתמונות הישרות הקוים כאשר הוצאנו בם ממרכזיהם אל זויותיהם הקוים אשר תארנו יותכו כלם אל משולשים |
| It has now been clarified that the triangle is a root and a beginning of all the shapes. | הנה כבר התבאר שהמשולש שרש והתחלה לכל התמונות כלן |
The Discussion of the Triangular Numbers, their Production and their Sides |
הדבור במשולשים המספריים וצמיחתם וצלעותיהם |
| The triangular numbers are sums of the natural numbers beginning from the one. | ואמנם המשולשים הם הוספות המספרים הטבעיים אשר התחלתם האחד קצתם על קצת |
|
הנה האחד כאשר נרשם הנה הוא משולש בכח |
|
וכאשר נוסיף על האחד השנים היו השלשה ראשית המשולשים בפעל |
|
עוד תוסיף שלשה אשר ימשכו לשנים על המשולש הראשון בפעל ויהיה המשולש השני בפעל והוא ששה |
|
עוד תוסיף ארבעה ויהיה המשולש השלישי בפעל והוא עשרה |
| So on, the production of the triangles is generated from the addition of the natural numbers to each other. | עוד כן תתחדש תולדת המשלשים בתוספת המספרים הטבעיים קצתם אל קצתם |
| From what you observe in this diagram, the triangular numbers are produced from the addition of the natural numbers to each other. | וממה שתבחנהו בזאת הצורה שהמספרים המשולשים יתילדו מחבור המספרים הטבעיים קצתם על קצת |
|
כמו ג' שתשא חבור ב' וא' |
|
א' ג' ו' י' ט"ו כ"א |
| The production of the sides of these triangles is as the production of the natural numbers according to their succession: | ואמנם התילד צלעות אלו המשולשים הוא כפי התילד המספרים הטבעיים על המשכם |
|
הנה יהיה צלע הראשון בכח האחד |
|
וצלע הראשון בפעל ב' |
|
וצלע השני בפעל ג' |
|
וצלע השלישי בפעל ד' |
| In this way, each side of the triangles is produced from the production of the natural numbers. | ובזה הצד מהתילד המספרים הטבעיים יתילד כל צלע מצלעות המשולשים |
|
וזה כאשר נתחיל להניח האחד תחלה |
|
עוד יתילד כל מספר מהמספרים הטבעיים בפני עצמו בטורים ימשכו קצתם לקצת תחת האחד כפי מה שתראה מצוייר בזאת הצורה |
|
המשולש הראשון בכח אחד וצלעו אחד |
|
והמשולש הראשון בפעל שלשה וצלעו השני' |
|
והמשלש השני בפועל ששה וצלעו שלשה |
|
והמשלש השלישי בפעל עשרה וצלעו ארבעה |
|
והמשלש הרביעי בפעל ט"ו וצלעו חמשה |
| What should be examined concerning these triangular numbers is that each triangle among them encompasses the geometrical triangles by a number that is equal to the number of the preceding square, i.e. preceding by one rank, and so on always. | ומה שראוי שתבחנהו באלו המספרים המשולשים שכל משולש מהם יקיף מהמשלשים המדותיים במניין שוה למספר המרובע הקודם ר"ל במדרגה אחת וכן תמיד |
The Discussion of the Square Numbers, their Sides and their Production |
הדבור במרובעים המספריים וצלעותיהם והתילדם |
| The regular square shapes are not produced as the triangles are produced from the three. | ואמנם התמונות המרובעות הישרות לא יתילדו כמו שיתילדו המשולשות מן השלשה |
| This is because they have four sides and four angles. | וזה שהן בעלות ארבע צלעות וארבע זויות |
| Such as: 4; 9; 16; 25; 36 | כמו ד' ט' י"ו כ"ה ל"ו |
| The shapes of these numbers are quadruple and equilateral, according to this example, i.e. the one illustrated at the end of the chapter, which is the diagram of all regular squares. | ורשמי אלו המספרים על הרבוע והשווי יהיה כפי זה המשל ר"ל המצוייר בסוף זה השער והיא התמונה אשר עליה יהיו המרובעים השוים תמיד |
| It is clear from the illustrated example below that the sides of the square numbers in their succession increase according to the root of the production of the natural numbers: | ומבואר נגלה מהדמיון המצוייר למטה שצלעות המרובעים המספריים כפי המשכם יתוספו כפי שרש צמיחת המספרים הטבעיים |
|
כי צלע הראשון בכח אשר הוא האחד אחד |
|
וצלע הראשון בפעל ב' |
|
וצלע השני בפעל ג' |
|
וצלע השלישי בפעל ד' |
| And so is their production always. | וכן צמיחתם תמיד |
| It is already said in the first chapter how the equilateral numbers are produced, as it was mentioned there. | וכבר אמרנו במאמר הראשון איך יתילדו המספרים השוי הצלעות למה שקרה שם זכרו |
| Yet, it is necessary to return to discuss it, since this is the place of its particular mention. | ואמנם מחוייב להשיב המאמר עליו הנה אחר שהוא מקום זכרו המיוחד בו |
| It is said that the production of the square numbers is from the successive addition of the natural odd numbers. | ונאמר שצמיחת אלו המרובעים מהוספת הנפרדים הטבעיים קצתם על קצת |
|
אחר שהתחלתם האחד אשר הוא נפרד בכח ומרובע בכח |
|
כי השלשה אשר הם נפרד ראשון כאשר נוספו על האחד היה הגעת זה ארבעה והוא המרובע הראשון בפעל |
|
וכאשר נוסף על המרובע הראשון בפעל הנפרד השני אשר הוא חמשה היה הגעת זה תשעה והוא המרובע השני בפעל |
| Since it was already mentioned once, there is no need to elaborate about it more than by saying that the production of the equilateral squares is by adding the natural odd numbers to each other. | ולפי שאנו כבר זכרנו זה פעם אחת אין צורך לנו להאריך ביותר מאשר נאמר שהולדת המרובעים השוי הצלעות אמנם תהיה בתוספת הנפרדים הטבעיים קצתם על קצת |
| Each side of any square among them is a root of that square, i.e. when it is counted by the measure of the units that are in it, the result is that square. | וכל צלע מצלעות כל מרובע מהם הוא שרש אותו המרובע ר"ל כאשר ימנה בשעורי מה שבו מהאחדים היה כללו הגעת אותו המרובע |
| When the roots of these squares are set successively, they accord with the order of the succession of the natural numbers. | וכאשר הונחו שרשי אלו המרובעים ילוו קצתם לקצת היו על סדר המספרים הטבעיים ימשכו קצתם לקצת |
| The rule of this discussion is in accordance with this. | וכלל זה המאמר על זה |
| This is the diagram of the squares, which was intended to be brought at the end of the discussion: | וזה צורת המרובעים אשר יעדנו להביאה בסוף הדברים |
|
המרובע הראשון בכח הוא האחד הנרשם בשחרות בזוית העליונה מצד הימין והוא הנרשם עליו א' |
|
והמרובע הראשון בפעל הוא המתקבץ מזה האחד ומשלשת האחדים האדומים אשר ילוו עליהם על תכונת תמונת הרושם |
|
והמרובע השני הוא המתקבץ מזה המרובע הראשון בפועל ומחמשת אחדים |
|
והמרובע השלישי בפעל הוא המתקבץ מזה המרובע השני בפעל ומשבעה האחדים האדומים הנלוים עליהם על תכונת תבנית הרושם |
|
והמרובע הרביעי בפעל הוא המתקבץ מזה המרובע השלישי בפעל ומתשעת האחדים השחורים הנלוים עליהם על תכונת תבנית הרושם |
| It should be examined that the geometrical shapes in this diagram are [the sums of] the natural odd numbers, according to their succession in the natural order. | וממה שראוי לך שתבחנהו שתמונות הרושם בזאת הצורה הם הנפרדים הטבעיים כפי המשכם בסדר הטבע |
The Discussion on the Pentagonal Numbers, their Production and their Sides |
הדבור במחומשים המספרים והתילדם וצלעותיהם |
| The pentagonal number, i.e. all pentagonal numbers, their production is not through the production of the triangle and the square, since the pentagon has five sides and five angles, such as: 1, 5, 12, 22, 35, 51, and the descriptions of these numbers by the quintuple and equality are as drawn at the end of this chapter. | ואמנם המספר המחומש ר"ל כלל המחומשים המספרים אין צמיחתם בצמיחת המשלש והמרובע כי המחומש לו חמשה צלעות וחמשה זויות כמו א' ה' י"ב כ"ב ל"ה נ"א ורשמי אלו המספרים על החמוש והשווי הם כפי מה שציירתי לך בסוף זה השער |
|
הנה צלע המחומש הראשון בפעל והוא ה' שנים |
|
וצלע המחומש השני בפעל והוא י"ב שלשה |
|
וצלע המחומש השלישי בפעל והוא כ"ב ארבעה |
| It is necessary that the sides of these pentagons follow each other as the sequence of the natural numbers, as clarified concerning the triangular and the square numbers. | וכן יחוייב שיהיו צלעות אלו המחומשים נמשכים יחד על סדר המספרים הטבעיים כפי מה שהתבאר במשולש והמרובע |
| The production of the pentagonal numbers: | ואמנם התילד המחומש |
|
הנה כבר התבאר כי אחר שהיה המחומש הראשון בפעל ה' והשני י"ב והשלישי כ"ב שאשר הוספנו אותו על המחומש הראשון אשר הוא א' בכח ארבעה עד שהיה המחומש הראשון בפעל |
|
וכאשר הוספנוהו על הראשון בפעל והוא ה' ז' עד שהיה י"ב והוא השני בפעל הנה מותר השבעה אשר הוספנום לסוף על הארבעה אשר הוספנום ראשונה שלשה |
|
והמחומש השני בפעל י"ב והשלישי בפועל כ"ב |
|
ואשר הוספנוהו על השני בפועל עד שיהיה השלישי בפעל עשרה ועשרה יותר משבעה שלשה |
| Hence, it is clear that the production of the pentagonal numbers is always by adding three to what was added to the preceding pentagonal number, and so it will be found always. | הנה אם כן כבר התבאר שתולדת המחומשים בתוספת שלשה לעולם על מה שהוספנוהו במחומש אשר לפניו וכן ימצא תמיד |
|
כי הנה המחומש הרביעי ל"ה ול"ה יותר מכ"ב בתוספת י"ג וי"ג יותר מי' שלשה |
| Thus, it is clear how the pentagonal numbers are produced. | הנה אם כן כבר התבאר איך יתילדו המחומשים |
| Furthermore, it will be clear from all that is said, how the hexagonal and the heptagonal numbers and the others are composed: | וגם כן כבר יתבאר מכל מה שאמרנו איך יתרכבו כמו כן המשושים והמשובעים וזולתם |
|
וזה שאנו כבר בארנו שהמשלשים יתחדשו בתוספת המספרים הטבעיים קצתם על קצת והמספרים הטבעיים יתוספו באחד אחד |
|
ובארנו גם כן כי המרובעים יתחדשו בתוספת המספרים הנפרדים קצתם על קצת והנפרדים יתוספו שנים שנים |
|
ובארנו שהמחומשים יתחדשו בתוספת המספרים אשר יתוספו קצתם על קצת שלשה שלשה |
|
ונאמר שהמשושים יתחדשו בתוספת המספרים אשר יתוספו קצתם על קצת ארבעה ארבעה |
|
והמשובעים יתחדשו בהוספת המספרים אשר יתוספו קצתם על קצת חמשה חמשה |
| So also for the octagonal and the nonagonal numbers, or whatever one wishes of these, they are generated as this drawn description. | וכן המשומין והמתושע או מה שתרצה מזה אמנם יתחדש בזה התאר המצוייר |
| Examples were already given for the pentagonal, hexagonal, heptagonal, and octagonal numbers. | הנה כבר המשלנו דמיונים למחומשים ולמשושים ולמשובעים ולמשומנים |
|
והבדלנו בחלוק שני גוונים המספרים אשר בם יוסיף כל מספר משוטח מהם על אשר לפניו |
| These numbers were assumed as an image of geometrical shapes, in order that what was mentioned will be clear, that the shapes of each species of the plane equi-angular numbers are produced from the addition of numbers that exceed [one another] by one number that never changes forever. | והנחנו אותם המספרים כדמות תבניות הרושם כדי שיתבאר בם מה שזכרנו מאשר כל מין ממיני המספרים המשוטחים בעלי הזויות השוות אמנם יתילדו תמונותיו בתוספת מספרים יעדיפו במספר אחד לא ישתנה לעולם אל בלתי תכלית |
|
אמנם המרובעים המספרים אשר יתוספו בם ר"ל מספרי התמונות אשר ישלימו אותם על גבולי תמונות הרושם תהיה העדפתם שנים שנים |
|
ואמנם המחומשים הנה העודף הנזכר בם יהיה שלשה שלשה |
| Likewise, for any rank shifted forward in the ranks of the species of the plane numbers, the excess mentioned exceeds by one. | וכמו כן כל מה שתעתיק מדרגה אחת במדרגות מיני המספרים המשוטחים יוסיף היתרון הנזכר באחד |
| משולש ח | משולש ז | משולש ו | משולש ה | משולש ד | משולש ג | משולש ב | משולש א | ||||||||||||||||||||||||||||||||||||||||
|
|
|
|
|
|
|
א | ||||||||||||||||||||||||||||||||||||||||
| מרובע ח | מרובע ז | מרובע ו | מרובע ה | מרובע ד | מרובע ג | מרובע ב | מרובע א | ||||||||||||||||||||||||||||||||||||||||
|
|
|
|
|
|
|
א | ||||||||||||||||||||||||||||||||||||||||
| מחומש ח | מחומש ז | מחומש ו | מחומש ה | מחומש ד | מחומש ג | מחומש ב | מחומש א | ||||||||||||||||||||||||||||||||||||||||
|
|
|
|
|
|
|
א | ||||||||||||||||||||||||||||||||||||||||
| משושה ח | משושה ז | משושה ו | משושה ה | משושה ד | משושה ג | משושה ב | משושה א | ||||||||||||||||||||||||||||||||||||||||
|
|
|
|
|
|
|
א | ||||||||||||||||||||||||||||||||||||||||
| triangle 8 | triangle 7 | triangle 6 | triangle 5 | triangle 4 | triangle 3 | triangle 2 | triangle 1 | ||||||||||||||||||||||||||||||||||||||||
|
|
|
|
|
|
|
1 | ||||||||||||||||||||||||||||||||||||||||
| square 8 | square 7 | square 6 | square 5 | square 4 | square 3 | square 2 | square 1 | ||||||||||||||||||||||||||||||||||||||||
|
|
|
|
|
|
|
1 | ||||||||||||||||||||||||||||||||||||||||
| pentagon 8 | pentagon 7 | pentagon 6 | pentagon 5 | pentagon 4 | pentagon 3 | pentagon 2 | pentagon 1 | ||||||||||||||||||||||||||||||||||||||||
|
|
|
|
|
|
|
1 | ||||||||||||||||||||||||||||||||||||||||
| hexagon 8 | hexagon 7 | hexagon 6 | hexagon 5 | hexagon 4 | hexagon 3 | hexagon 2 | hexagon 1 | ||||||||||||||||||||||||||||||||||||||||
|
|
|
|
|
|
|
1 | ||||||||||||||||||||||||||||||||||||||||
The Discussion about how All Plane Polygonals Consist of and are Resolved into Triangles |
הדבור באשר כל המשוטחים בעלי הזויות השוות מהמשולשים יתרכבו ואליהם יותכו |
| The explanation of what was said before, that the polygonal numbers are composed of the triangles and are resolved into them, follows from this. | וכבר קרה מזה ביאור מה שאמרנו לפנים שבעלי המספרים הישרי הקוים השוי הזויות הם מורכבים מהמשולשים ונתכים אליהם |
|
וזה שכבר נראה לנו שהמרובעים אמנם חודשו מהרכבת המשולשים |
|
כאשר הנחנו המשולשים על סדר כמו א' ג' ו' י' ט"ו |
|
עוד חברנו המשלש הראשון בכח אל המשלש הראשון בפעל והוא ג' יחודש המרובע הראשון בפעל והוא ד' |
|
וכאשר חברנו המשולש הראשון בפעל אשר הוא ג' אל השני בפעל אשר הוא ו' יתחדש המרובע השני בפעל אשר הוא ט' |
|
וכן כאשר חברנו המשולש השני בפעל והוא ו' אל המשולש השלישי בפועל והוא י' יחודש המרובע השלישי והוא י"ו |
|
וכן תמצא זה תמיד מתרכב מכל שני משולשים מהם מרובע כפי זה הסדר אשר המשלנו |
|
ובהפך זה המאמר יותך כל מרובע מהמרובעים אל המשולשים אשר מהם הורכב |
|
כי הארבעה אשר הוא מרובע יותך אל אחד ושלשה והם שני משולשים |
|
והתשעה אל שלשה וששה הם שני משולשים |
|
הנה כבר התבאר איך יתרכבו המרובעים מן המשולשים ויתכו אליהם |
|
ואמנם המחומשים כפי מה שאתאר וזה שהם יתרכבו מהמשולשים והמרובעים |
|
כאשר סדרנו המשולשים בטור כמו א' ג' ו' י' ט"ו |
|
עוד נסדר המרובעים בטור כמו ד' ט' י"ו כ"ה ל"ו |
|
עוד נחבר כל מדרגה עם נכחה |
|
הנה יתקבץ מחבור המשלש הראשון בכח אשר הוא א' עם המרובע הראשון בפעל אשר הוא ד' המחומש הראשון בפעל אשר הוא ה' |
|
וכן תמצאהו תמיד כאשר תרכיבהו כפי זה הסדר |
|
ובהפך זה המאמר יתבאר שהמחומשים יותכו אל המשולשים ואל מה שיורכב מהמשולשים ר"ל המרובעים |
|
כי החמשה יותך אל אחד וארבעה |
|
והי"ב אל שלשה ותשעה |
|
וכן מה שאחר זה |
|
הנה כבר התבאר איך יתרכבו המחומשים מהמשולשים ויותכו אליהם |
|
וכן תמצא גם כן שיתילדו המשושים מהרכבת המשולשים עם מה שיהיה נכחם בטור המחומשים כאשר הורכבו על דמיון מה שבארנו |
|
אלא שיהיה לעולם ראש הנחת המשולשים המשולש הראשון בכח אשר הוא אחד וראש מדרגות מספרי השטחים האחרים הראשון מהם בפעל |
|
והמשושה הראשון בפעל אמנם יחודש מהרכבת המשלש הראשון בכח אשר הוא האחד עם המחומש הראשון בפעל אשר הוא חמשה |
|
והמשושה השני מהרכבת המשלש הראשון בפעל אשר הוא ג' עם המחומש השני בפעל אשר הוא י"ב |
|
וכן המשובע גם כן יתחדש הראשון ממנו בפעל מהרכבת המשלש הראשון בכח אשר הוא א' עם המשושה הראשון בפעל |
|
והמשובע השני בפעל מהרכבת המשולש הראשון בפעל עם המשושה השני בפעל |
| So, all of them - the octagons, the nonagons and the other polygons - are always found, their production is from the triangles and they resolve into the triangle. | וכן ימצאו כלם תמיד המשומנים והמתושעים וזולתם מבעלי הזויות ותולדותם מן המשולשים והתכתם אל המשולש |
| To explain it to the sense [i.e. visually] we set a diagram of parallel lines [i.e. table]: the first line to the triangles; the second to the squares; the third to the pentagons; the fourth to the hexagons; the fifth line to the heptagons; and the sixth line to the octagons. | ולבאר זה לחוש נניח לו צורה מטורים נכחים הטור הראשון למשולשים והשני למרובעים והשלישי למחומשים והרביעי למשושים והטור החמישי למשובעים והטור הששי למשומנים |
| Whoever wants to add numbers of surfaces to this diagram should stick to the order we relied on and aimed at. | ומי שירצה להוסיף בזאת הצורה מספרים מבעלי השטחים ידבק בסדור אשר נשעננו וכוננו בו אליו |
| What is clear from this diagram is that: | וממה שיתבאר בזאת הצורה |
| י | ט | ח | ז | ו | ה | ד | ג | ב | א | מספר הצלעות | מספר השטחים | א |
| נה | מה | לו | כח | כא | טו | י | ו | ג | א | המשולשים | א | ב |
| ק | פא | סד | מט | לו | כה | יו | ט | ד | א | המרובעים | ב | ג |
| קמה | קיז | צב | ע | נא | לה | כב | יב | ה | א | המחומשים | ג | ד |
| קצ | קנג | קכ | צא | סו | מה | כח | טו | ו | א | המשושים | ד | ה |
| רלה | קפט | קמח | קיב | פא | נה | לד | יח | ז | א | המשובעים | ה | ו |
| רפ | רכה | קעו | קלג | צו | סה | מ | כא | ח | א | המשומנים | ו | ז |
| 10 | 9 | 8 | 7 | 6 | 5 | 4 | 3 | 2 | 1 | no. of sides | surface no. | 1 |
| 55 | 45 | 36 | 28 | 21 | 15 | 10 | 6 | 3 | 1 | triangles | 1 | 2 |
| 100 | 81 | 64 | 49 | 36 | 25 | 16 | 9 | 4 | 1 | squares | 2 | 3 |
| 145 | 117 | 92 | 70 | 51 | 35 | 22 | 12 | 5 | 1 | pentagonals | 3 | 4 |
| 190 | 153 | 120 | 91 | 66 | 45 | 28 | 15 | 6 | 1 | hexagonals | 4 | 5 |
| 235 | 189 | 148 | 112 | 81 | 55 | 34 | 18 | 7 | 1 | heptagonals | 5 | 6 |
| 280 | 225 | 176 | 133 | 96 | 65 | 40 | 21 | 8 | 1 | octagonals | 6 | 7 |
|
שהמינים הראשונים בפעל ר"ל המשוטחים המספריים יעדיפו על המשכם ברחב הצורה במשלש הראשון בכח והוא האחד |
|
והמינים השניים בפעל יעדיפו על המשכם ברחב הצורה במשולש הראשון בפעל ר"ל בשלשה |
|
והמינים השלישים בפעל יעדיפו על המשכם ברחב הצורה במשולש השני בפעל |
|
וכן המינים הרביעים בפעל יעדיפו על המשכם במשלש השלישי בפעל |
|
וכן מה שאחר זה |
| The discussion on the plane polygonal numbers is complete. | וכבר נשלם המאמר במספרים המשוטחים בעלי הזויות |
The Discussion on the Solid Numbers |
הדבור במספרים הגרמיים |
| The distinction between the solid and the surface has already been explained in the previous discussion | כבר בארנו במה שקדם מן המאמר מה ההפרש בין הגרם והשטח |
| It was said that the solid adds a dimension of depth to the surface, since the surface has only length and breadth | ואמרנו שהגרם הוא אשר יוסיף משך בעומק על השטח אחר שהשטח אורך ורחב לבד |
|
ואמרנו עומק או גובה הכל שוה |
| People have said it all before. | וכבר אמרו כל זה אנשים |
| Since the discussion on triangles preceded in the section on surfaces, as it was more worthy of precedence due to the reason we indicated: that they are a principle and a root for all surfaces, the pyramids precede in the discussion on solid numbers. | ואחר שהיה קודם במאמרנו על השטח הדבור על משולשים לעלה אשר תארנו מהיותר ראוי לקדימה אחר שהם פנה לכל השטחים ושרש יהיה הקדימנו המאמר הזה בגרמים על המזונבים |
| By pyramids I mean those that begin in a rectilinear plane base, rising in height, until they culminate in one. | ורצוני במזונבים אשר יתחילו מתושבת משוטח ישר הקוים ר"ל הצלעות עוד יעלו בגובה עד שיכלו אל האחד |
| All the surfaces of this shape are triangles except its base, since the bases of the pyramid species are either triangles, or squares, or pentagons, or hexagons, or others of the mentioned surfaces, but its [other] surfaces are triangles only. | כי זאת התמונה אם שיהיו שטחיה כלם משלשים מלבד תושבתה כי התושבות לזה המין המזונב כבר יהיו משולשים או מרובעים או מחומשים או משושים או זולת זה מהשטחים אשר זכרנו ואמנם בשטחיו כאשר היה מזונב הם משולשים לבד |
| Since the pyramid whose bases are all triangles, i.e. the triangular pyramid is a principle and a root for all the pyramids, as they are composed from it and dissolve into it, it is necessary to begin with its description. | ומפני שהמזונב אשר תושבותיו יחד משולשים ר"ל הגרם המשולש פנה ושרש לכל הגרמים בעלי הזויות אחר שהרכבתם ממנו והתכתם אליו יחוייב שנתחיל בו לתארו |
| This solid is encompassed by four triangles. | ונאמר שזה הגרם יקיפו בו ארבעה משולשים |
|
כי אלו ידמה מדמה מהתמונות הגרמיות מהמדתיות משולש שוה הצלעות בעל שלשה המשכים שוים באורך לצלעותיו יצאו מזויות תושבתו עוד יפגשו על נקדה נכחית למרכז בנפילת עמוד יהיה כבר ידומה גרם מזדנב לו ארבעה שטחים שוים אחד מהם התושבת והשלשה הנשארים המקיפים בו |
|
וכמו כן גם כן אלו ידמה מדמה מרובע שוה יצאו מזויותיו ארבע צלעות כל אחד מהם לצלע מצלעות תושבתו ויפגשו כלם על נקדה נכחית למרכז בנפילת עמוד המרובע ידומה גרם המזדנב ר"ל מחודד לו חמשה שטחים כמו זאת הצורה אחד מהם שטח מרובע והוא תושבתו והארבעה הנשארים משולשים והם המקיפים בו |
|
וגם כן אלו ידמה מדמה כמו זה במחומש והמשושה והמשובע וזולתם ימצא בכל אחד מהם המשכים יצאו מזויותיהם ויפגשו על נקדה לנגד מרכזיהם יחדשו באותם הקוים היוצאים מהזויות ותכליות תושבתם משולשים ויהיה הגרם מזדנב ר"ל מחודד |
| If wishing to examine it, one can look at the examples drawn previously in the chapter of the discussion about the triangle as a principle for all the two-dimensional regular surfaces. | וכאשר תרצה לבחון זה עיין בדמיונים המצויירים קודם זה בשער הדבור על שהמשולש פנה לכל בעלי הזויות והקוים הישרים מהשטחים המדותיים |
| Again, it is said that the production and increase of every absolute number, as the line, is from the one, such as 1, 2, 3, 4, 5, 6, to that which is added infinitely. | ונשוב עתה ונאמר שכל מספר מוגבל כמו הקו הנה תולדתו וצמיחתו מהאחד וזה כמו א' ב' ג' ד' ה' ו' אל מה שיתוסף מזה תמיד |
| From the linear numbers the angled surfaces and solids are composed, except that they are not produced and composed accidentally from the construction in thought, but according to a definition and order, as revealed previously when defining the production of the surfaces. | ומאלו המספרים הקויים מה שיתרכבו בעלי הזויות מהשטחים והגרמים אלא שלא יתילד ויתרכב איך שיזדמן מההרכבה במחשבה אבל על גבול וסדר כמו שכבר גלינו במה שקדם בהגבילנו צמיחת השטחים |
| The production of the solid numbers and their growth | ואמנם התילד המוגשמים וצמיחתם |
|---|---|
|
הנה המשלש המזדנב ר"ל הגרם המשלש התילדו בהנחת האחד בגובה עוד תניח תחתיו המשולשים המספריים אשר כבר זכרנום כל אחד תחת אשר לפניו במדרגה וסדר כפי המשכם ונפעל זה תמיד מה שנרצה להוליד המזדנבים כי יתחדש גוף מחודד משולש |
|
ואמנם המרובעים המזדנבים ר"ל המחודדים הנה הם יתחדשו כאשר תסדר אותם השטחים המרובעים המספריים אשר זכרנום לפנים והנחת האחד ראשון להם עוד תשים המרובעים תחתיו ימשכו קצתם לקצת יתחדש מזה מרובע מזדנב |
| Likewise for the pentagonal pyramid, the hexagonal pyramid, the heptagonal pyramid, and what is similar to these. | וכן המחומש והמשושה והמשובע ומה שידמה זה |
|
הנה הגרמיים המזדנבי' המשולשים ר"ל המחודדים יהיו מדרגות חדושם כפי משך אלו המספרים א' ד' י' כ ל"ה נ"ו |
|
הנה כל אחד מאלו המספרי' אמנם יתילד מסדר המספרים קצתם תחת קצת מאחד האחד עוד האחד עד אשר תרצה |
|
ואמנם האחד הנה הוא גרם המחודד המשולש בכח |
|
ואמנם הארבעה הנה הוא השיני והוא הרכבת שני משולשים האחד והשלשה |
|
ואמנם העשרה הוא מהרכבת שלשה משולשים אחד ושלשה וששה |
|
וכן הנשארים כפי זה הסדר נמצא בזה התאר |
|
ואמנם מדרגות התחדש הגרמים המרובעים המחודדים כפי המשכם הנה על משך אלו המספרים א' ה' י"ד ל' נ"ה |
|
כי כל אחד מאלו המספרים אמנם התילד מהרכבת המרובעים הפשוטים אשר אמרנו עליהם לפנים קצתם על קצת כמו שאמרנו במשולש המחודד |
| As described in this example, so are the pentagonal pyramid, the hexagonal pyramid and others. | וכפי זה המשל אשר תארנו יהיה המחומש המחודד והמשושה וזולתם |
| It is clarified from all that was noted, that the units that are in each of the sides of the pyramids are equal to the number of the surfaces arranged in them, whatever they may be, from the one to the surface of the base. | ומבואר מכל מה שזכרנו שכל צלע מצלעות מנין המחודדים יחד שוה מה שבם מהאחדים למנין השטחים המסודרים בם היו מה שהיו מהאחד עד שיגיע אל שטח התושבת |
| It should not be left out from all that was mentioned, that the simple triangle is the principle and the root of all the surfaces. | ולא יעלם ממה שזכרנו שהמשולש הפשוט הוא הפנה והשרש לכל השטחים |
| The triangular pyramid is the principle of all pyramids. | והמשולש המוגשם המחודד הוא הפנה לכל המחודדים |
| After mentioning the pyramids among the solids, now the one that is called truncated is discussed, which is every angular solid, whose arrangement and construction are according to the arrangement of the construction of the pyramids, except that its height does not end in one, which is the apex of the pyramid, and the angles of its top base are equal in number to the angles of its bottom base, this species is called the truncated. | ואחר שכבר הבאנו בזכירת המזונב מהמוגשמים נדבר על אשר נקראהו מחוסר והוא כל גשם בעל זויות היה בסדורו והרכבתו על סדר הרכבת המחודדים אלא שלא יכלה בגבהו אל האחד אשר הוא עליון שבמחודד והיו זויות שטח עליונו שוות במספר לזויות שטח תושבתו הנה זה המין יקרא המחוסר |
|
וזה אם היה חסר מהמחודד באחד לבד אשר הוא עליון המחודד יקרא מחוסר אחד |
|
ואם היה חסר מהאחד שטח אחד יקרא מחוסר שנים |
|
ואם היה חסר שטח שלישי יקרא מחוסר שלשה |
|
וכן המחוסר ארבעה אם היה חסר ארבעה שטחים |
| So it is always found. | וכן תמצאהו תמיד |
| Since the production of this truncated solid is already clarified, as there is no difference between it and what was already explained before about the construction of the pyramid, except that a surface or surfaces are subtracted from the apex of the pyramid, which is the one, this is whole of the discussion. | ואחר שכבר התבאר איך צמיחת זה הגשם המחוסר אחר שאין הפרש בינו ובין מה שבארנו לפנים מהרכבת המוגשם המחודד אלא שאנו נחסרהו מעליון המחודד אשר הוא האחד שטח או שטחים הנה זה כלל המאמר על זה |
| This is the time to begin the discussion on the rest of the aforementioned solids, which are the cubes, the bricks, the wedges, the circulars, the sphericals, the scalene, and the heteromecic, while endeavoring to explain it, with the help of God. | ואמנם המאמר על שאר הגרמים אשר זכרנום לפנים והם המעוקבים והלבנים והאריחיים והקשתיים והכדוריים והמתחלפי הצלעות והזולתיים באורך הנה זה עת להתחיל בו ונשתדל לפרשו בג"ה |
The Discussion on the Cube Number |
הדבור במספר המעוקב |
| The square equilateral plane numbers discussed above, such as 1, 4, 9, 16, 25, 36, when each one of these surfaces is composed with a surface similar to it in quantity, so that the number of the surfaces composed with each other is as the units of the length and the breadth of the base surface, it is called a cube number. | ונאמר עתה שהמספרים המרובעים השוים המשוטחים אשר דברנו עליהם לפנים והם כמו א' ד' ט' י"ו כ"ה ל"ו כאשר הורכב כל אחד מאלו השטחים לדומה לו מהשטחים בכמות עד שיהיה מספר השטחים המורכב קצתם על קצת כמו אחדי אורך ורחב שטח התושבת יקרא מעוקב |
| The rule of the discussion: the cube is that whose length, breadth and depth are equal to each other. | וכלל המאמר כי המעוקב הוא אשר ארכו ורחבו ועמקו שוה קצתו לקצתו |
|
כי הארבעה אשר הם מספר שוה כל צלע ממנו שנים כאשר הורכב עליהם ארבעה אחדים היה כלל שמנה ושמנה שנים בשנים עוד בשנים וזה שוה המרחקים בשלשה ר"ל האורך והרחב והעמק |
|
וכן גם כן התשעה אשר כל צלע מצלעיותיו שלשה כאשר הרכבו עליהם שני שטחים אחרים שוים להם היה הכלל כ"ז הוא הגעת מוכה ג' בג' עוד בג' |
| So for the rest of the cube numbers according to this description. | וכן שאר המספרים המעוקבים כפי זה התאר |
| Their general rule: each number is multiplied by the measure of its units, then the product is multiplied again by the measure of the units of the first number, so the total sum is a cube number. | והכלל הכולל בהם שכל מספר נכפל בשעור אחדיו עוד נכפל הכל בשעור אחדי המספר הראשון שנית הנה הכלל יהיה מספר מעוקב |
| From these it is known that any plane square is a surface that has four angles and four sides. | הנה כבר קרה מזה שנדע שכל מרובע פשוט הוא שטח ולו ארבע זויות וארבע צלעות |
| Any cube has six equal surfaces, eight equal angles, and twelve equal sides. | וכל מעוקב לו ששה שטחים שוים ושמנה זויות שוות ושתים עשרה צלעות שוות |
| It is said that the sides, the angles, the surfaces, and the lines of the cube are in a harmonic proportion. | ואמנם מה שנאמר בו כי צלעות המעוקב וזויותיו ושטחיו וצלעותיו ר"ל קוויו ביחס חבוריי |
|
וזה שיחס השטחים והם ששה אל הזויות והם שמנה ביחס המוסיף שליש |
|
ויחס הזויות והם שמנה אל הצלעות והם י"ב ביחס המוסיף חצי |
|
הנה יחס השטחים והם ששה אל הצלעות והם שתים עשרה ביחס הכפל השניי |
| These two ratios, i.e. the superparticular ratio and the multiple ratio, are the species of the simple ratios, as already clarified above. | ושני אלו היחסים ר"ל המוסיף חלק והכפל הם מיני היחסים הפשוטים החבורים כמו שכבר התבאר זה לפנים |
| Hence, the solid number whose dimensions are equal, i.e. the length, breadth and depth, is necessarily the cube number. | הנה אם כן המספר הגרמי השוה המרחקים ר"ל האורך והרחב והעומק הוא המעוקב בהכרח |
The Discussion on the Scalene Numbers |
הדבור במספרים הגרמיים המתחלפי המרחקים השלשה |
| Now we should discusses the solid that is opposed to the cube, that is, the unequal in all dimensions, i.e. whose length differs from its breadth, and both together differ from its height. | וממה שראוי שנדבר עתה הוא בגרם אשר יקביל המעוקב והוא המתחלף המרחקים יחד ר"ל אשר ארכו מתחלף לרחבו והם יחד מתחלפים לגבהו |
| For example: breadth = 2, length = 2, height = 4; or length = 3, breadth = 4, depth = 5. | משל זה שיהיה הרחב ב' והאורך ג' והגובה ד' או יהיה האורך ג' והרחב ד' והעומק ה' |
| Or other modes of diversity of the excess of some sides over others. | או זולת זה מאופני החלוף בתוספת קצת הצלעות על קצת |
| The rule of this discussion is that none of its dimensions is equal to another dimension. | וכלל המאמר שלא יהיה דבר ממרחקיו שוה למרחק אחר ממנו |
| This number is called by many names: | וזה המספר יקרא בשמות רבים |
|
מהם שאנשים יקראוהו הסולמיי מפני שיעלה בהוספה מרחבו לארכו ומארכו לגבהו |
|
ויקראהו אחרים הארחיי להתדמות לאריח שהוא גוף מתחלף השטחים בשלשת מרחקיו |
| This is the image of their shape and their order according to their natural succession: | וזה לך דמיון תמונתם וסדורם כפי המשכם בסדר הטבע |
|
תניח לוח הולך ברחב ר"ל רחב הלוח נרשם מחולק במדרגותיו מדרגות המספרים כפי סדרם בטבע |
|
ונניח תחתיו לוח שני יותר חסר ממנו במדרגה מצד התחלת המדרגות עוד נעמיד בכל מדרגה ממנו מה שיתקבץ מהכאת אשר למעלה ממנו מהלוח הראשון עם אשר לפניו |
|
ותניח גם כן תחתיהם לוח שלישי כמו הלוח השני וכפי מנין מדרגותיו ונעמיד בו תחת כל מדרגה מהלוח מה שיתקבץ ממדרגה אשר עליה ומהכאתה במדרגה השלישית אשר עליה מהלוח הראשון עד שישלם בזה הטור השלישי ויהיו מדרגותיו כלם הם מספרי הגרמים הסולמיים כפי המשכם בטבע מבלתי שיחטא בם באחד מהם בפנוי ב' ממדרגותיהם |
| Examine it in this diagram written here: | ותבחן זה בזאת הצורה אשר רשמנו בכאן |
| יא | י | ט | ח | ז | ו | ה | ד | ג | ב | א |
| קי | צ | עב | נו | מב | ל | כ | יב | ו | ב | |
| תתקץ | תשך | תקד | שלו | רי | קכ | ס | כד | ו |
| 11 | 10 | 9 | 8 | 7 | 6 | 5 | 4 | 3 | 2 | 1 |
| 110 | 90 | 72 | 56 | 42 | 30 | 20 | 12 | 6 | 2 | |
| 990 | 720 | 504 | 336 | 210 | 120 | 60 | 24 | 6 |
The Discussion on the Parallelepipedon Numbers |
הדבור במספרים הגרמים הזולתיים |
| Since these two species, i.e. the cube and the scalene numbers correspond to the parallelism of the two extremes, as the cube is equal in its three sides, and the other is unequal in its three sides, the mean between these two are the solid numbers that are called parallelepipedons, i.e. their surfaces are heteromecic. | ואחר שהיו שני אלו המינים ר"ל המעוקב והמתחלף הצלעות יוקבלו בהקבלת שני הקצוות אחר שהמעוקב שוה הצלעות השלשה והאחר מתחלף הצלעות השלשה הנה האמצעי בין שני אלו הם המספרים הגרמיים אשר יקראו הזולתיים האורך ר"ל אשר פשוטיהם זולתיי האורך |
| As will soon be stated, the heteromecic number is the number whose measure is defined in a plane as a quadrangular right-angled shape, whose breadth is shorter than its length by one, such as: 2, 6, 12, 20, 30, 42 and their similar. | הנה המספר הזולתי האורך כבר יאמר שהוא המספר אשר ירשם שעורו בפשוט רושם מרובע נצב הזויות ורחבו פחות מארכו אחד כמו ב' ו' י"ב כ' ל' מ"ב והדומה להם |
| When each of these heteromecic surfaces is given a height that is same as one of its sides - the parallelepipedon numbers are produced. | הנה כאשר שמנו לכל אחד מאלו הפשוטים הזולתיי האורך גובה באחד מצלעותיו יתילדו מזה המספרים הגרמיים הזולתיים |
| This is an image of their formation: | וזה לך דמיון הנחתם |
|
שני טורים מהם הראשון והשני הם משלשת הטורים הקודמים בשער הוצאת המספרים הגרמיים הסולמיים |
|
ונכה כל מדרגה ממדרגות הטור השני מהם בכל אחת מאשר עליה בטור הראשון ר"ל אשר עמדה מהכאת אחת מהן באחרת |
|
ונעמיד שני הכללים בשתי מדרגות נבדלות הקטנה מהן קודמת לגדולה ושתיהן תחת המדרגה הנעשית בהכאה מהטור השני |
|
וכאשר תשלים הלוח בזאת המלאכה הנה כבר שמת מדרגות הטור השלישי כפל מדרגות הטור השני |
| Thus, all parallelepipedon numbers are reproduced according to their natural succession, none of them is missing. | וכבר העמדנו בו כל המספרים הגרמים הזולתיים כפי המשכם בסדר הטבע לא יבצר מהם אחד |
| י | ט | ח | ז | ו | ה | ד | ג | ב | א | |||||||||
| צ | עב | נו | מב | ל | כ | יב | ו | ב | ||||||||||
| תתק | תתי | תרנב | תקעו | תמח | שצב | רצד | רנב | קף | קנ | ק | פ | מח | לו | יח | יב | ד | ב | |
| 10 | 9 | 8 | 7 | 6 | 5 | 4 | 3 | 2 | 1 | |||||||||
| 90 | 72 | 56 | 42 | 30 | 20 | 12 | 6 | 2 | ||||||||||
| 900 | 810 | 652 | 576 | 448 | 392 | 294 | 252 | 180 | 150 | 100 | 80 | 48 | 36 | 18 | 12 | 4 | 2 | |
If some of the sides exceed some of them by more than one, i.e. by two, or three, or four, such as  , ,  , or , or  , or more than that, it is not called heteromecic number but an oblong number. , or more than that, it is not called heteromecic number but an oblong number.
|
ואמנם אם יוסיפו קצת הצלעות על קצת ביותר מאחד ר"ל שנים או שלשה או ארבעה כמו שנים בארבעה וארבעה בששה או חמשה בשבעה או מה שלמעלה מזה לא יקרא מה שהוא כן זולתיי האורך אבל יקרא המוסיף האורך |
| Know that two of the quadrangular surfaces of the parallelepipedon numbers are parallel squares and the rest of their surfaces, i.e. the four remaining surfaces of any of the solid numbers among them, each of them is a heteromecic number, its four sides are equal, of one measure, and its eight sides are equal, of one measure differing from the measure of the four by one, additional or deficient. | ודע שהמספרים הגרמיים הזולתיים יהיו לעולם שני שטחים משטחיהם מרובעים מקבילים ושאר שטחיהם ר"ל הארבעה שטחים הנשארים בכל מספר גרמיי מהם הנה כל אחד מהם יהיה זולתיי האורך ויהיו ארבעת צלעותיו שוים על שעור אחד ושמנה מצלעותיו שוים על שעור אחד מתחלף לשעור הארבעה באחד אם מוסיף ואם חסר |
The Discussion on the Natural Root of the Numerical “Sameness” and Numerical “Otherness” |
הדבור בשורש הטבעיי להוא הוא והזולתיות המספריים | ||||||||||||||||||||||||||||
| The "same" is the number, whose breadth is has the value of its length | אמנם ההוא הוא הנה הוא המספר אשר רחבו הוא ארכו בהגעה | ||||||||||||||||||||||||||||
| The root of the "sameness", according to the ancients of the pythagoreans, is 1, since when it is multiplied by 1, the length is the breadth, and the product is each of them not parting from them at all. | ואמנם היה שרש ההוא הוא אצל הקדמונים מכת פתאגורוש האחד לפי שהוא כאשר יוכה באחד היה האורך הוא הרחב והמתקבץ הוא כל אחד מהם לא יצא מעצמו כלל | ||||||||||||||||||||||||||||
| The square numbers are called "same", as they partake in this conception, since their length is their breadth. | עוד נקראו המספרים המרובעים הוא הויים ללקחם הדמוי מזה לפי שארכם הוא רחבם | ||||||||||||||||||||||||||||
| The more appropriate among the numbers to be the root of the otherness is the one which does not differ from the above mentioned true fundamental "same", except by one. | והיה היותר ראוי מהמספרים להיות שרש לזולתיי הוא אשר לא ישתנה מההוא הוא האמתי השרשיי הנזכר לפנים אלא באחד | ||||||||||||||||||||||||||||
| Therefore, the root of the "other" numbers should be the second number | הנה בראוי אם כן יהיה שרש המספרים הזולתיים הוא המספר השניי | ||||||||||||||||||||||||||||
| According to this the "same" and the "other" are drawn. | הנה כפי זה היו מקישים ההוא הוא והזולת | ||||||||||||||||||||||||||||
It is already clear from what we preceded and explained:
|
וכבר היה שתבאר ממה שהקדמנו ובארנו שכל נפרד הנה האחד מיוחד לו אחר שבין שני חלקיו האחד לעולם | ||||||||||||||||||||||||||||
|
בהכרח הם יעדיף אחד משני חלקיו לאחד | ||||||||||||||||||||||||||||
|
ושכל זוג הנה השניות מיוחד לו אחר שיחלק בשני חלקים דומים נמשלים | ||||||||||||||||||||||||||||
| It should be said that the odd number, as it is the root of the "same", is particular for the nature of the "same". | ובראוי נאמר כי הנפרד מיוחד אחר שזאת שהוא שורש ההוא הוא מיוחד לו לטבע ההוא הוא | ||||||||||||||||||||||||||||
| And the even number is particular for the "other". | והזוג מיוחד לטבע הזולת | ||||||||||||||||||||||||||||
| Since, from the successive addition of the odd numbers, beginning with the one, the "same" numbers are generated, i.e. the squares, that are equal by the measure of the length and the breadth, as explained a few times. | כי בתוספת הנפרדים קצתם על קצת ובהקדים האחד בהנחה ראשונה להם יצמחו מספרי ההוא הוא ר"ל המרובעים השוים במרחק האורך והרחב כמו שבארנו זה פעמים | ||||||||||||||||||||||||||||
| And from the successive addition of the natural even numbers, beginning with the two, the "other" numbers are produced. | ובתוספת הזוגות הטבעיים קצתם על קצת ובהקדים השנים ראשונה להם בהנחה יתילדו הזולתיים | ||||||||||||||||||||||||||||
|
ואמנם קראנו אלו המספרים השוים באורך וברחב מספרי הוא הוא ללקחם דמוי הוא הוא האחד | ||||||||||||||||||||||||||||
|
וקראנו המתחלפים באורך וברחב באחד זולתיים ללקחם דמוי השניות היוצא מטבע ההוא הוא האמיתי באחד | ||||||||||||||||||||||||||||
| When comparing the terms of the "same" numbers and the terms of the "other" numbers in two lines, each term compared to its corresponding, one finds that the excess of the "other" term over the corresponding "same" rank is the number, from which the name the term is derived. | וכאשר תקביל בשני טורים בין מדרגות המספרים ההוא הויים ומדרגות המספרים הזולתיים כל מדרגה במקביליה תמצא מותר המדרגה הזולתיית על הנכחיית לה ההוא הויית הוא המניין אשר ממנו נגזר שם המדרגה | ||||||||||||||||||||||||||||
|
|
The Discussion on the Brick Numbers |
הדבור על המספרים הלבניים והמגודרים |
| The numbers that are similar to bricks are of a species of the parallelepipedon numbers, in which the measure of their four equal sides is one less than the measure of their eight equal sides. | ואמנם המספרים הדומים ללבנים הוא המין מהמספרים הגרמיים הזולתיים אשר יהיה שעור צלעותיו הארבעה השוים פחות אחד משעור צלעותיו השמנה השוים |
| Those are the terms whose explanation is written in the column of the parallelepipedon numbers. | והם המדרגות הנרשם עליהם באורם בטור המספרים הגרמיים והזולתיים |
| These are those whose length and breadth are equal and the height is one less than each of them. | והם אשר ארכם ורחבם שוה ויחסר הגובה מכל אחד מהם אחד |
| If its height exceeds the length and breadth that are equal by the excess of the "other", i.e. by one, or by the excess of the oblong number, i.e. by more than one, such numbers, one of whose three dimensions exceeds the others, are called beam solids. | ואמנם אם יוסיף בגובה על האורך והרחב השוים תוספת הזולתיי ר"ל אחד או תוספת המוסיף באורך ר"ל יותר מאחד היו מה שהיה המספר הנוסף באחד מהמרחקים השלשה הנה כל אלו יקראו מוגשמים אריחיים |
| Such as:
|
כמו ג' בג' בד' |
|
|
או ה' בה' בו' |
| Or as much as one wishes, as long as the height is longer than both the length and the breadth. | או כמה שתרצה אחר שיהיה הגובה יותר ארוך מכל אחד מהאורך והרחב |
The Discussion on the Circular and Spherical Numbers |
הדבור במספרים הסבוביים והכדוריים |
| After describing the quality of the solids whose surfaces are of straight lines: | ואחר שכבר הבאנו תאר הגרמים בעלי השטחים הישרי הקוים |
| We say, as we said that the cube number is of equal dimensions, i.e. the length, the breadth, and the depth, it is divided into two parts: | הנה נאמר כמו שאמרנו שהמספר המעוקב הוא השוה המרחקים ר"ל האורך והרחב והעומק וזה יחלק לשני חלקים |
|
אם שיהיה המספר אשר הוא שרש כל אחד משטחיו נזכר בכלל השטח עוד נזכר בכלל הגרם חוזר על עצמו עוד נזכר גם כן בכלל הגרם כאשר הוכה בו פעם או שני פעמים או יותר מזה |
|
ואם שלא יהיה כן |
| Those, whose roots are expressed in their total among these cube numbers are called spherical numbers. | הנה אשר שרשיהם נזכרים בכללם במבטא הגעתם מאלו המספרים המעוקבים הם אשר יקראו המספרים הכדוריים |
| Since they revolve, turning back as a sphere, to the number from which they begin. | אחר שיסבבו מתהפכים כמו הכדור אל המספר אשר ממנו התחלתם |
| Such as the cube number, whose side is 5 or 6, for as much as one multiplies one of them by the same, and the product by its root, it is as described. | כמו המספר המעוקב אשר הצלע מצלעותיו חמשה או ששה כי כל מה שתכה אחד משני אלו בכמהו עוד מה שיתקבץ בשרשו יהיה נמצא כמו שתארנו |
|
וזה שאנו אם נכה חמשה בחמשה היה המתקבץ כ"ה וישוב החמשה מבוטא בו בכלל וישוב אליו |
|
עוד נכה החמשה גם כן בכ"ה ותמצא החמשה גם כן מבוטא בו בכללם והוא קכ"ה |
|
|
וכן אם תכה הקכ"ה בחמשה |
|
|
ומה שהתקבץ גם כן בחמשה |
|
תמצאהו בזה התאר לעולם |
|
וכן גם כן הששה בזה הענין משפטם משפט החמשה |
|
וכן תמצא זה באחד כי האחד בכמהו |
|
עוד בכמוהו עוד בכמהו עוד כן תמיד בלתי יוצא מעצמו אבל סובב על עצמו |
| Since what was described is already clear, it is said that the circular number is formed by multiplying the number once by itself: | ואחר שכבר התבאר מה שתארנו נאמר שהמספר הסבוביי מזה שתכה המספר פעם אחת בעצמו |
|
|
כמו א' בא' |
|
|
וה' בה' |
|
|
וו' בו' |
| If it is multiplied more than once, it is a spherical number. | ואם הוכה יותר מפעם הנה הוא מספר כדורי |
| Since the circles are surfaces, and the spheres are solids. | אחר שהעגולים פשוטים והכדורים גרמיים |
| This is the end of the discussion concerning the solid numbers. | וזה סוף המאמר על המספרים הגרמיים |
The Discussion of the Indications of the Ancients that the Beginning of Numbers is similar to the Beginning of the World |
הדבור ברמזי הראשונים על ההתחלות המספריות הדומות להתחלות העולם |
| From what was said before about the "other" numbers follows their difference from the heteromecic numbers. | וכבר ימשך למה שאמרנו לפנים על המספרים הזולתיים ההפרש בם מהמספרים המוסיפים באורך |
| For we already explained that the "others" are those, either of whose dimension exceeds the other by one. | שאנו כבר בארנו כי הזולתיים הוא אשר אחד ממרחקיו השנים יותר מהאחר באחד |
| While the heteromecic is that whose production accords with this aspect, but the excess of one of its dimension over the other is greater than one. | ואמנם המוסיף הוא מה שהיתה צמיחתו כפי זה הצד אלא שתוספת אחד ממרחקיו על האחר יותר מאחד |
|
כמו ח' המתקבץ מהכאת ב' בד' |
|
|
וכמו י"ח אשר יתקבץ מהכאת ג' בו' |
| And those similar to this, where the difference between the two numbers, one of which is multiplied by the other, is more than one. | והדומה לזה ממה שהחלוף בו בין שני המספרים אשר יוכה אחד מהם באחר ביותר מאחד |
| He [= Nicomachus] said: because of the difference between these numbers, i.e. the "same", i.e. those that have root and the "others", i.e. those whose length exceeds over their breadth by more than one, the separation, the division, and the divergence were created in the number of the species. | אמר ולחלוף אלו המספרים ר"ל ההוא הויים ר"ל השרשיים והזולתיים ר"ל אשר יוסיף ארכם על רחבם יותר מאחד חודשה ההפרדה וההחלק והסתעפות מה שבמספר מהמינים |
| He [= Nicomachus] said: it is appropriate that the ancients began the principles of number by starting the discussion about the nature of the world. | אמר ובראוי מה שהתחילו הקדמונים הראשיות במספר בהתחילם המאמר על טבע העולם |
| Plato mentioned that the nature of the world is of three ends: | אם אפלאטון הנה זכר שטבע העולם משלשה גבולים |
|
אחד מהם ההוא הוא |
|
והשני הזולת |
|
והשלישי מהעצם אשר אינו מתחלק |
| This statement, may God lead you straight, is far from injustice and of very subtle property. | וזה המאמר יישירך השם רחוק העול מאד דק הענין |
| Plato refers in it to the principles of the world and this is a thing that is beyond the art of number. | ואפלאטון ירמז בו אל ראשיות העולם וזה דבר חוץ ממלאכת המספר |
| Had I not agreed not to mix anything into the science of this book, I would have passed over this chapter, and had I not been sure that it would not be conceived as useless lengthiness, and had I not been confident in your current level in philosophy, I would not have mentioned to you that there is a hint under this discussion, and not that it is far from injustice and of very subtle property. | ולולא שאני הסכמתי שלא אערב דבר בחכמת זה הספר עברתי זה השער ולולא שאני בטוח שלא ידומה עלי לאריכות לבלתי תועלת וגם בטחוני במדרגתך היום מהעיון לא זכרתי לך שתחת זה המאמר רמז ולא שהוא רחוק העול ודק הענין |
| The author of this book [= Nicomachus] said: Philolaus says that all the things that exist are necessarily either limited, or limitless, or limited and limitless together. | אמר מניח הספר אמנם פילולאוש יאמר שכל הדברים אשר הם נמצאים הנה הם בהכרח אם מוגבלים נכללים ואם בלתי מוגבלים ואם מוגבלים ובלתי מוגבלים יחד |
| It is said: and this third saying is about the manner of the number and its image. | אמר וזה המאמר השלישי אמנם הוא מאופן המספר ודמיונו |
| For the number is composed of the units, and its species are even and odd, which indicate the equality and inequality, the "sameness" and "otherness", the bound and boundlessness, the definite and indefinite | כי המספר מורכב מהאחדים ומיניו זוג נפרד והם מורים על השווי והחלוף וההוא הויים והזולתיים וההקפה ובלתי הקפה וההגדרה ובלתי הגדרה |
| What was said is clear. | ויהיה מה שנאמר מבואר |
| He said that the things that exist are produced and persist by the opposite of different and similar, and what was shown about the composition and harmony is appropriate, since the composition is doubtlessly, as explained in the introduction of the first section of this book, from similar diverse things, as the composition is a mixture of things of diverse definitions and similar sepcies. | ואמר שהדברים אשר הם נמצאים התילדו ועמדו מהמתנגדים המתחלפים הדומים ובראוי מה שהראו החבור וההסכמה כי החבור אמנם יהיה בלי ספק כמו שבארנו בפתיחת המאמר הראשון מזה הספר מהדברים המתחלפים הדומים הנמצאים כי החבור אמנם הוא ערוב דברים מתחלפי הגדרים דומי הסוגים |
| Abū Yusūf said: he epxressed this statement and compared the limited things to the numbers, which have roots, that are limited, since their root is expressible, that they are formed after it is counted by it self; and he compared the unlimited to the "other" things that are mute, and their root is inexpressible. | אמר אבו יוסף אמנם אמר זה המאמר והוא ימשיל המוגבל מהדברים במספרים הנגדרים שהם מוגבלים אחר שידובר בגדרם ר"ל שרשם שאחר שימנה בעצמו יחודש ויראו וימשיל הבלתי מוגבלים מהדברים הזולתיים שהם אלמים לא ידובר בשרש דבר מהם |
| He meant by limited and unlimited together the heteromecic, for among them are those that have roots as well as those that have no root. | ורצה במוגבל ובלתי מוגבל יחד המוסיף האורך כי ממנו שרשי וממנו בלתי שרשיי |
| Those that have a root are limited and those that have no root are unlimited. | הנה השרשיי מוגבל ובלתי שרשיי בלתי מוגבל |
Properties of the species of Numbers and their Mutual Harmony |
הדבור בקצת סגולות מיני המספרים ומה שיראה בהתילדם והקבלת קצתם לקצת |
| We have already brought in the introductory comments what we wanted to preface. | כבר הבאנו בהקדמות מה שרצינו להקדימו |
| Now, it will be shown how many of those described in the description of the "same" and "other" numbers are produced: | ונראה איך יתילדו הרבה ממה שתארנו מתואר המספרים ההוא הויים והזולתיים |
|
וזה בשנסדר המספרים המרובעים אשר נולדו בהוספת הנפרדים הטבעיים קצתם על קצת ר"ל הנגדרים בטור |
|
עוד נסדר הזולתיי האורך ר"ל אשר התילדו מתוספת הזוגות המתילדים קצתם על קצת בטור שני |
| For this way of action is truly seen visible in the nature of all. | כי זה האופן מן הפועל באמת כבר נראהו גלוי בטבע הכל |
| The line according to this example is: | ויהיה הטור כפי זה המשל |
| קמד | קכא | ק | פא | סד | מט | לו | כה | יו | ט | ד | א | |
| קנו | קלב | קי | צ | עב | נו | מב | ל | כ | יב | ו | ב | |
| התוספת | יב | יא | י | ט | ח | ז | ו | ה | ד | ג | ב | א |
| 144 | 121 | 100 | 81 | 64 | 49 | 36 | 25 | 16 | 9 | 4 | 1 | |
| 156 | 132 | 110 | 90 | 72 | 56 | 42 | 30 | 20 | 12 | 6 | 2 | |
| addition | 12 | 11 | 10 | 9 | 8 | 7 | 6 | 5 | 4 | 3 | 2 | 1 |
| It is clear from the wonders of the nature's action that the first of the "others" is in the double ratio to the first of the "same"; the second to the second are in the sesquialter ratio; the third to the third are in the sesquitertian ratio; the fourth to the fourth are in the sesquiquartian ratio; and so on, one always finds them together according to this description. | והוא מבואר מפליאות פועל הטבע שהראשון מהזולתיים ביחס הכפל השניי אצל הראשון מן ההוא הויים וכשני אל השני ביחס המוסיף חצי והשלישי אל השלישי ביחס המוסיף שלישי וכן הרביעי אל הרביעי ביחס המוסיף רביע וכן תמצאם תמיד יחד בכמו זה התאר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||
| What was said afore is also clarified in these two lines, that if the excess of each term of the "others" over its counterpart among the "same" is taken and set in a third line successively, the terms are found to be the numbers by the natural succession. | והתבאר גם כן בשני אלו הטורים מה שכבר אמרנו במה שקדם שאנו אם לקחנו תוספת כל מדרגה מהזולתיים על נכחה מהמדרגות ההוא הויים והנחנוהו בטור שלישי כפי המשכיה מצאנו מדרגות זה הם המספרים על סדר הטבע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||
This is found also by:
|
וכן תמצאהו כאשר תתחיל במדרגות ההוא הויים ותשים ראש מדרגותיהם ארבעה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד תוסיף תוספת כל מדרגה מהם על נכחה ממדרגות הזולתיי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עוד תסדרם בטור שלישי כפי המשכיהם תמצא זה בסדר המספרים הטבעיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כי תוספת ד' על ב' ב' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותוספת ט' על ו' ג' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן תמצאהו תמיד בזה התאר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If you take their ratio, if they accord with this assumption and you wish to take their ratio, you will find them in the same ratios by which they were seen previously: | ואמנם אם תקיש ביניהם והם כפי זאת ההנחה ותרצה ליחסם תמצאם באותם היחסים אשר נראו בראשונה בעצמם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה שד' אצל ב' בייחסם הכפל השניי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וט' אצל ו' ביחס המוסיף חצי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וי"ו אצל י"ב ביחס המוסיף שליש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכמו זה תמצאהו תמיד על צד הענין הראשון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||
| It is clear that the excess of the "same" to each other are the natural odds | ואמנם תוספת מדרגות ההוא הויים קצתם על קצת הוא מבואר שהם הנפרדים הטבעים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||
| and the natural odds and natural evens and the multiple ratio and the superparticular ratio [apparently broken sentence] | והנפרדים הטבעיים והזוגות הטבעיים ויחס הכפל ויחס המוסיף חלק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואמנם אם תניח הראשון מהזולתיים אמצעי בין שני הראשונים מן המרובעים ר"ל שנים בין אחד וארבעה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותניח השני מן הזולתיים אמצעי בין אשר אחריהם מן המרובעים ר"ל ו' בין ד' וט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן השלישי הזולתיי בין שני המרובעים אחרים אחר אשר הנחת | ||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן תעשה כל מדרגה ממדרגות הזולתיי כפי המשכם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תמצא זה ביחס מדרגות זוג הזוג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שעור הראשון אצל השני כשעור השני אצל השלישי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן תמצא כל שלשה מספרים מהם ר"ל מאלו אשר הממצע בין כל שתי מדרגות מההוא הויים מהם מדרגה מהזולתיים כפי סדרם אשר בארנו בזה היחס ר"ל ששעור הראשון מן השני כשעור השני מן השלישי ובהפך גם כן השלישי אצל השני כשני אצל הראשון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומבואר שאלו המדרגות כאשר היו כפי זה הסדר לא יהיה תוספת ממדרגותיהם קצתם על קצת שוים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה צורתו |
| פא | עב | סד | נו | מט | מב | לו | ל | כה | כ | יו | יב | ט | ו | ד | ב | א |
| 81 | 72 | 64 | 56 | 49 | 42 | 36 | 30 | 25 | 20 | 16 | 12 | 9 | 6 | 4 | 2 | 1 |
| The property of terms of the even-times-odd numbers is that when they are arranged successively in a special line, they are in the succession of the natural numbers. | ואמנם זוג הנפרד הנה סגולת מדרגותיו בסדורם כפי המשכם בטור מיוחד סדור המספרים הטבעיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| That is, the excesses of the terms of the even-times-odd numbers one over the other are equal: | וזה שזוג הנפרד הגעות תוספת מדרגותיו קצתם על קצת שוים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כי תוספת י' על ו' כמו תוספת י"ד על י' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן תוספת ב' על א' כמו תוספת ג' על ב' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וישיג שני אלו יחד שיהיה האמצעי נכפל כמו שני הקצוות מקובצים וכבר בארנו זה ודומה לו במאמר הראשון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The triangular numbers are generated from the combination of the "same" and the "others", each term with its counterpart, successively. | ונאמר שהמספרים המשולשים אמנם יתחדשו מהרכבת ההוא הויים והזולתיים כל מדרגה עם נכחה על משך מדרגותיהם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כי המדרגה הראשונה מההוא הויים והוא האחד כאשר הורכבה עם המדרגה הראשונה מהזולתיים והוא ב' יחודש המשולש הראשון בפעל והוא ג' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר תרכיב המדרגה הראשונה מהזולתיים והיא ב' עם המדרגה השנית מהוא הויים והוא ד' יחודש המשולש השני בפעל והוא ו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן תמצא הרכבת אלו המדרגות בזה התאר תמיד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| It is visible from what was described and explained before, that when one of the dimensions of the square becomes different from the other by one, whether by addition to it or by subtraction from it, it becomes an "other". | ויראה ממה שתארנו ובארנו קודם שהמרובע כאשר יתחלף באחד משני מרחקיו לאחד באחד אם בתוספת עליו ואם בחסרון ממנו יצא אל הזולתיי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Hence, the rank of the square that is equivalent to the "other" is as the rank of equality to the aforementioned five relations, since one of the two numbers exceeds the other and the other is less than it and the equality is a foundation for them. | הנה אם כן מדרגת המרובע השוה לזולתיי כמדרגת השוה להקשות החמש אשר הקדמנו זכרם אחר שאחד משני המספרים נוסף על האחר והאחד חוסר ממנו והשווי פינה להם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| It was also said that the true "same" is the one, and the true "other" is the duality. | וכבר אמרנו גם כן שההוא הוא האמתי הוא האחד והזולתיי האמתי הוא השניות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| In addition, the odd is similar to the "same" and the even to the "other", therefore, the regular square is similar to the "same", since it consists of the odds, and the [heteromecic number], whose two dimensions differ by one, is similar to the "other", since it consists of the evens. | עוד אחר זה כבר ידמה הנפרד להוא הוא והזוג לזולת ולזה בעצמו כבר ידמה המרובע השוה ההוא הוא אחר שהוא מורכב מהנפרדים וידמה המתחלף שני המרחקים באחד הזולתיי אחר שהוא מורכב מהזוגות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Returning to what was mentioned about that which is evident concerning these two species, i.e. the "same" and the "other", when they are arranged successively as aforementioned, which is the succession of the two species together in one line, so that their terms are such that each term of one of the two species is between two terms of the other species successively. | אמנם עתה נשוב אל מה שזכרנוהו ממה שיראה משני אלו המינים ר"ל ההוא והזולתיי כאשר יסודר כפי הסדר אשר זכרנוהו לפנים והוא סדר שני המינים יחד בטור אחד בסדר שיהיו מדרגותיהם כן כל מדרגה מאחד משני המינים בין שתי מדרגות מהמין האחד על המשך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כמו א' ב' ד' ו' ט' וכן תמיד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| One finds that every three terms of this line, when it is arranged by this order, when they are equal in ratio, they differ in their additions to each other; and when they are equal in their additions to each other, they differ in their ratio. | הנה זה הטור כאשר יסודר זה הסדר תמצא כל שלשה מדרגות מהם כאשר השתוו ביחס יתחלפו בהגעות תוספותיהם קצתם על קצת וכאשר ישתוו בהגעות תוספות קצתם על קצת יתחלפו ביחס | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כי א' ב' ד' ביחס הכפל השניי אבל תוספת ד' על ב' ב' ותוספת ב' על א' א' הנה היחס אחד והתוספות מתחלפות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמנם שלשה המדרגות אשר הן ב' ד' ו' תוספת ו' על ד' כמו תוספת ד' על ב' אלא שהיחס יתחלף לפי שיחס ו' אל ד' בלתי יחס ד' אל ב' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן גם כן שלשת המדרגות אשר הן ד' ו' ט' שהן ביחס אחד ואין התוספות שוות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן גם כן שלשת מדרגות ו' ט' י"ב בהגעות התוספות מתחלפות ביחס | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן תמצא כל שלשה מדרגות מזה הטור המורכב מן ההוא הויים והזולתיים כפי ההרכבה אשר תארנו לפנים בזה התאר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| It is clarified from what was mentioned, that when the "other" and the "same" are such that the two dimensions of the "other" differ so that the length exceeds the breadth by one, for every three terms of this line that are matching in ratio, but differ in the excesses, the difference of the excesses is also one. | ויתבאר ממה שזכרנו שכאשר היה הזולתיי וההוא הוא אלו ששני מרחקי הזולתיי יתחלפו בתוספת אחד באורך על הרחב שיהיו כל שלשה מדרגות מזה הטור שיסכימו ביחס ויתחלפו בהגעת התוספת שיהיה חלוף התוספות גם כן באחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כי ב' אשר הוא המותר בין ד' וב' יוסיף על האחד אשר הוא המותר אשר הוא בין א' וב' אחד וכן תמיד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| We further say what is strengthened by what was mentioned before, that the odds verify more strongly the nature of the "same", i.e. those that have roots: | ונאמר עוד שממה שיחזק עם מה שזכרנוהו קודם כי הנפרדים יותר חזקי ההאמתה בטבע ההוא ר"ל הנגדרים מה שאנחנו זוכרים אותו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה שאנו נסדר המספרים אשר התחלתם מהאחד והמשכם ביחס הכפל השניי בטור כמו א' ב' ד' ח' י"ו ל"ב ס"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונסדר גם כן המספרים אשר התחלתם מהאחד והמשכם על יחס הכפל השלישיי כמו א' ג' ט' כ"ז פ"א רמ"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| One finds that the odd terms in each of the two lines are of the "same", i.e. necessarily having root, i.e. the terms that are in a place whose number is odd, their values have roots; those that are in a place whose number is even, their values do not have roots. | ונמצא מדרגות הנפרדים מכל אחד משני הטורים מספרים הוא הויים ר"ל שרשיים בהכרח ר"ל שהמדרגות אשר תהיינה במקום נפרד ממנין המדרגות הגעותיהם נגדרות ואשר הם במקום זוג ממנין המדרגות הגעותיהם בלתי נגדרות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| It is further said that the solid cube numbers, since they are also similar to the "same", as their dimensions are equal, they are also produced from the odd numbers. | ונאמר עוד שהמספרים הגרמיים המעוקבים אחר שהם גם כן דומים להוא הויים לפי שמרחקיהם שוים הנה הם יתילדו מהנפרדים גם כן | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| This is found by the natural wonderful technique that is easy to execute: | וימצא זה בזאת התחבולה הנפלאה בטבע הנקלה במעשה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה שאנו נסדר הנפרדים הטבעיים בטור | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וימצא המעוקב הראשון בכח אשר הוא האחד הוא הנפרד הראשון בכח אשר הוא א' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והמעוקב השני בכח הוא ח' מהרכבת שתי מדרגות ימשכו לאחד מהנפרדים והם ג' וה' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והמעוקב השלישי אשר הוא כ"ז מהרכבת שלשה מדרגות ממדרגות הנפרדים ילוו לשתי המדרגות אשר הורכב מהם המעוקב השני והם ז' ט' י"א כי כל זה כ"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| So, one always finds, whenever skipping to a cube, that its combination is from odd terms, whose number are the same as the number of its place among the terms of the cubes, i.e. that the second cube consists of two odds, the third consists of three odds, and so on for what follows. | וכן תמצא זה תמיד כל מה שתדלג אל מעוקב תמצא כי הרכבתו ממדרגות נפרדים מנינם כמו מקומו ממנין מדרגות המעוקבים ר"ל כי המעוקב השני מהרכבת שני נפרדים והשלישי מהרכבת שלשה נפרדים וכן מה שאחר זה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Yet, for every cube, the first of the odds, from which it is composed, follows the end of the terms, from which its preceding cube is composed, and the terms are taken successively by the order of the natural odd numbers. | אלא שכל מעוקב הנה ראש הנפרדים אשר מהם יורכב אחר סוף מדרגה מהמדרגות אשר הורכב מהם המעוקב אשר לפניו עוד המדרגות לקוחות על משך סדר הנפרדים הטבעיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| This is the rule of the discussion about it, and its diagram is as you see: | הנה זה כלל המאמר על זה וצורתו כמו שתראה |
| מא | לט | לז | לה | לג | לא | כט | כז | כה | כג | כא | יט | יז | טו | יג | יא | ט | ז | ה | ג | א |
| המעוקב הששי | המעוקב החמישי | המעוקב הרביעי | המעוקב השלישי | המעוקב השני | המעוקב הראשון | |||||||||||||||
| ריו | קכה | סד | כז | ח | א | |||||||||||||||
| 41 | 39 | 37 | 35 | 33 | 31 | 29 | 27 | 25 | 23 | 21 | 19 | 17 | 15 | 13 | 11 | 9 | 7 | 5 | 3 | 1 |
| sixth cube | fifth cube | fourth cube | third cube | second cube | first cube | |||||||||||||||
| 216 | 125 | 64 | 27 | 8 | 1 | |||||||||||||||
[Relative Quantity] |
|
The Discussion on The Ten Means |
הדבור על האמצעיים העשרה |
| It is what the author of this book in Arabic calls al-ʽIyād. | וזה אשר יקרא אותו בעל זה הספר בערבי אלעיאד |
| Its meaning: a determination of two proportions or more between two given terms, | ומשמעותו הגבלת שני יחסים או יותר מזה בין גבולים מונחים |
|
אם מעצמם ר"ל שתהיה ההגבלה מוצאת מעצמי הגבולים עצמם קצת אל קצת |
|
ואם מיתרון קצתם על קצת |
|
ואם משני העניינים יחד |
| The proportion is the being of two determined terms given with respect to each other. | והיחס הוא ישות שני גבולים מוגבלים מונחים אחד מהם אצל האחר |
| This is at least a relation of three terms. | ובפחות מה שיהיה ההתיחסות בשלשה גבולים |
|
כמו ד' וב' וא' |
|
כי שעור ד' אצל ב' כשעור ב' אצל א' |
|
וכמו כן בהפך הנה ההגבלה הנקראת אלאיעד אשר בין א' וב' אצל ד' הוא יחסם אחד |
| This al-ʽIyād is essentially the given number itself. | וזה אלאיעד הוא בעצם המספר המונח עצמו |
| The numbers in this ratio can always be extended by [more] units, as explained before, more than once, in this book, so that the numbers will be four, or five, or six, or more, as one wishes. | וזה היחס כבר אפשר שיתוספו מספריו תמיד באחדות כאשר קדם פירושינו לו יותר מפעם בספרנו זה כמו שיהיו המספרים כאשר היו ארבעה או חמשה או ששה או יותר מזה כפי מה שתרצה |
|
כמו שתניח א' ב' ד' ח' י"ו ל"ב ס"ד כי אלו כלם ביחס הנזכר בין שלשת הגבולים תחלה |
| The proportion that is subject to addition: | ואמנם ההתיחס אשר יפול ביתרון |
|
כמו א' ב' ג' |
|
כי אין שעור האחד מב' כשעור ב' מג' |
|
אבל שעור תוספת ג' על ב' הוא השעור אשר בו יוסיף ב' על האחד וכן הוא גם כן בהפך |
| It is al-ʽIyād even if the given numbers are numerous: | וזה אלאיעד גם כן אם ירבו המספרים המונחים |
|
הנה כפי זה המשל ימצאו שעורי התוספות בחלוף כפי זה המשל גם כן כמו ב' וג' וד' וה' |
|
כי תוספת ה' על ג' כמו תוספת ד' על ב' וכן ימצא גם כן בהפך |
| It is said that the proportions that are acknowledged by all the ancients - Pythagoras, Plato and others - are three: | ונאמר שהאמצעיים אשר יודו בם כל הקדמונים ר"ל פיתאגורש ואפלאטון וזולתם הם שלשה |
|
האחד מהם לחשבון |
|
והשני למדות |
|
והשלישי לחבור הנגונים |
|
ולאמצעי המדות והחבור מקבילים אחרים שלשה |
|
לחבור הקבלה אחת |
|
ולמדות שתי הקבלות |
|
אלא שהם אינם בעלות שמות כמו אלו ונקראו מהמספר ונקראו רביעית וחמישית וששית |
| Four other proportions were added to these six proportions constituting the ten proportions, as the ten was perfect for Pytagoras. | וכבר אמרו אחר זה המאמר הקדום שהאמצעיים עשרה והוסיפו על אלו הששה ארבעה אחרים להשלים האמצעיים העשרה להיות לעשרה שלמות אצל פיתאגורש |
| We will discuss the description of these proportions: | ונדבר בתאר אלו האמצעיים |
The Arithmetic Proportion |
וניחד המאמר תחלה במצוע המספריי |
| The discussion about the priority of the number in the four sciences is already completed. | ונאמר הנה כבר נשלם מאמרנו בהיות המספר ראוי בקדימה באומניות הארבעה |
| The numbers given in the arithmetic proportion are according to the natural succession, or similarly to the natural succession. | ועם זה הנה המספרים המונחים לאמצעי החשבון הם כפי סדר הטבע או מה שהיה דומה לסדר הטבע |
| Hence it is necessary to precede this proportion to the other proportions, since it is antecedent to the other antecedent proportions and all the more so to their opposites. | הנה אם כן יחוייב בהכרח שנקדים זה האמצעי על שאר האמצעיים שאחר שהיה קודם על שאר האמצעיים הקודמים הנה יותר ראוי מזה שיקדם על המקבילים להם |
|
הנה האמצעי המספריי יהיה כאשר תניח שלשה מספרים או יותר מזה והונחו כפי חבור הטבע ימשכו קצתם לקצת |
|
כמו א' ב' ג' ד' ה' |
|
כי אלו המספרים בהכרח תוספת כל אחד מהם על מה שלפניו אחד |
| If one wishes to arrange them according to this ratio, which is equality of the difference: | ואם תרצה לסדרם בזה היחס אשר הוא שווי היתרון |
|
אתה תסדר המספרים הטבעיים בטור |
|
עוד תתחיל במספר אשר תרצה לשומו בגבול הראשון ותעיין כמה בינו ובין המספר אשר תרצה לשומו אמצעי מהמדרגות אשר על סדר הטבע וימשכו כמו אותו המנין מהמדרגות בין האמצעי והגבול השלישי וכן בין השלישי והרביעי או במה שתניח מהמדרגות בזה היחס |
|
כמו שתניח הגבול הראשון ב' והגבול השני ד' |
|
ואין ספק שתניח הגבול השלישי על דמיון מרחק ד' מב' והוא מרחק ו' מד' ר"ל על מרחק שתי מדרגות |
|
וכן אם תניח גבול רביעי היה הוא אשר ירחק מו' בשתי מדרגות והוא ח' |
| According to this way the terms are set. When you wish to increase them, their excess is always by the same addition. | וכפי זה הדרך תניח הגבולים כאשר תרצה להרבותם הנה תהיה הוספתם לעולם בתוספת אחד |
|
ובפרט זה האמצעי ר"ל אמצעי החשבון המתחייב לאלו בלתי זולתם שהגבול האמצעי מהם כשיכפל על ב' אם היו המדרגות המונחות להם אמצעי אחד ישוה שני הקצוות כאשר יקובצו |
|
אם היו המדרגות בעלות שני אמצעיים הנה קבוץ שני האמצעים כמו קבוץ שני הקצוות |
|
ולהם סגולה שנית גם כן והיא שיחס כל אחד אל עצמו כמו יחס מותרי הגבולים אלו על אלו קצתם על קצת |
|
עוד סגולה שלישית והיא היותר קשה ונעלמת מדעת רבים והיא כי כפילת שתי הקצוות האחד על האחר כאשר נערכהו אל כפילת האמצעי הוא יותר פחות בערך כפילת מותרי הגבולים אלו על אלו בין שיהיו הגבולים נפרדים במספרם ובין שיהיו איזה מספר שיהיה כאשר יקובצו היו יותר משני הקצוות יחד |
|
ולהם סגולה רביעית והיא ממה שזכרנוהו הראשונים מתאריהם והיא שיחס אשר בין הגבולים הקטנים מהם יותר גדול מיחס אשר בין הגבולים הגדולים |
|
כי שנים ושלשה וארבעה אשר הם בסדר זה האמצעי |
|
שעור ארבעה מהם והוא הגבול הגדול אצל השלשה כיחס המוסיף חלק השלישי |
|
ושעור שלשה אצל השנים והוא הגבול הקטן כיחס המוסיף חלק החציי |
|
וזה היחס גדול מהראשון כשעור תוספת החצי על השליש |
|
וזה יראה ביחס הניגוניי בהפך כי היחס אשר בין הגבולים הגדולים יותר גדול מהיחס אשר הקטנים |
|
מפני זה אמצעי המספר הם הפכים לאמצעי הנגון |
|
ואמצעי המדות הם אמצעיי בין אלו ההפכים כי יחס אשר בין הגבולים הגדולים הוא שוה ליחס אשר בין הקטנים |
| This is the end of the discussion of the arithmetic proportion. | זה הוא סוף המאמר על האמצעי המספרי |
The Geometic Proportion |
|
|
ואמנם האמצעי המדותיי הוא כאשר יהיו שלשה מספרים או יותר מזה והיה שעור הראשון מהם אצל השני כשעור השני אצל השלישי וכן בהפך |
|
כמו ד' ח' י"ו שהם בזה התאר בהתיחסות המדותיי |
| These given numbers in the geometric proportion differ from the given numbers in the arithmetic proportion, since these terms themselves are proportional and their differences between each other are unequal, while the differences of the former are equal and their terms are disproportional. | וכבר יתחלפו אלו המספריים המונחים לאמצעי ההנדסה למספרים המונחים באמצעי המספר לפי שאלו המדרגות עצמן מתיחסות ומותרי קצתם על קצת בלתי שוה ואותן היו מותריהם שוים ומדרגותיהם בלתי מתיחסות |
|
וסגלת זה האמצעי ההנדסיי כי למותרי הגבולים קצתם על קצת מהיחס כמו מה של גבולים עצמם מהיחס |
|
|
כי יחס מותרי י"ו על ח' והוא ח' אל מותרי ח' על ד' והוא ד' כמו יחס י"ו אל ח' וח' אל ד' |
|
וכן אם היו גם כן הגבולים ביחס הכפל השלישיי כמו ג' ט' כ"ז |
|
וכן הוא תמיד כל מה שתניח מן המדרגות באי זה מן היחסים החמש שתרצה אחר שיהיו הגבולים נמשכים ביחס והבן זה |
|
ולזה האמצעי סגולה שנית והוא שמוכה הגבול האמצעי בעצמו כמו שני הקצוות מוכה אחד מהם באחר |
| It should be noticed to what was said before, concerning the "same" and "other" numbers, that between each term of one of them there is a mean term of the other successively in the natural order. | וראוי שנשים לב למה שאמרנו לפנים שמספרי ההוא הוא והזולת בין כל מדרגה מאחד מהם ומדרגה מן האחר אמצעי על המשכם בסדר הטבע |
|
כמו ד' ו' ט' י"ב י"ו |
| Since every three [subsequent] terms among them [starting with as "same" number] are proportional. | כי כל שלשה מספרים יוקחו מהם מתיחסים |
| If their first term is "other" the differences are equal. | ואם יהיה הגבול הראשון מהם זולתיי יהיה שווי היתרון |
| The rule of this statement: between every two subsequent "same" numbers there is one "other" number that follows the same ratio with them, which is less[?] than for the cube "same" numbers. | וכלל זה המאמר שיפל בין כל שני מספרים הוא הויים נלוים מספר אחד זולתיי ימשך עמם על יחס בפחות מההוא הויים המעוקבים |
| Since between every two subsequent cubes among them there are two numbers that follow the same ratio. | כי בין כל שני מעוקבים נלוים מהם שני מספרים ימשכו עמם על יחס |
|
כי המעוקב הראשון בפעל ח' והמעוקב השני כ"ז ובין שני אלו המספרים המעוקבים שני מספרים והם י"ח וי"ב וכבר יתיחסו אלו הארבעה ביחס אחד |
| This is what was necessary to be said here. | וזה ממה שיחוייב שנאמר אותו הנה |
|
דע שכל מספר מרובע הוכה במספר מרובע הנה המקובץ מרובע |
|
וכל מספר מרובע יוכה במספר בלתי מרובע יהיה המתקבץ מספר מעוקב |
|
כמו ח' בכ"ז שהוא רי"ו כי זה מהכאות בו' עוד בו' |
|
ואם תכה מעוקב בזולתיי לא יתקבץ מעוקב |
|
כמו ח' בו' שהוא מ"ח ומ"ח אינו מעוקב ולא שטח נגדר |
|
ואם תכה מספר זולתיי במספר אחר זולתיי לא יחוייב שיתקבץ מרובע פשוט ולא גם כן מעוקב |
|
ואם תכה זוג בזוג יהיה המתקבץ זוג |
|
ואם תכה נפרד בנפרד יהיה מה שיתקבץ נפרד |
| The author of this book [= Nicomachus] said: Plato has already explained it in his book that is called in Greek language politeia. | אמר מניח הספר וכבר באר זה אפלאטון בספרו אשר יכונה בלשון יוני בולוטיא |
The Harmonic Proportion |
|
| The harmonic proportion is when there are three given numbers, which are not in one ratio to each other, as the terms in the geometric proportion, and the excesses of each over the other are not equal, as [those of] the terms in the arithmetic proportion, but the measure of the greater term to the measure of the smaller term is as the measure of the excess of the greater term over the mean term to the excess of the mean over the smaller.
|
ואמנם האמצעי החבוריי הנה הוא כאשר היו שלשה מספרים מונחים ולא יהיה לקצתם אל קצת יחס אחד כמו הגבולים המדותיים לא יתרוני קצתם על קצת שוים כמו הגבולים המספרים אבל יהיה השעור הגבול הגדול אצל שעור הגבול הקטן כשעור מותר הגבול הגדול על הגבול האמצעי אל מותר האמצעי על הקטן |
|
כמו ג' ד' ו' |
| Also, the measure of the greater term to the greater excess is as the measure of the smaller term to the smaller excess. | וכן גם כן שעור הגבול הגדול אצל גדול שני המותרים כשעור הגבול הקטן אצל קטן שני המותרים |
|
וסגולת אלו הגבולים הפכית לגבולי אמצעי החשבון והמדות יחד |
|
לפי שאלו הגבולים יחס הגבול הקטן מהם אל האמצעי גדול מיחס האמצעי אל הגדול |
|
וגבולי האמצעי החשבון יחס הקטן אל האמצעי פחות מיחס האמצעי אל הגדול |
|
ואמנם גבולי אמצעי המדות אם היו שלשה גבולים מהם מונחים כפי תנאם יהיו כלם ביחס אחד |
|
ולאמצעי חבוריי סגלה אחרת והיא שהכאת כל אחד משני הקצוות מגבוליו באמצעי מקובצים כמו כפל מה שיהיה משני הקצוות מוכה אחד מהם באחר |
[Extracting a Third Term in the Arithmetic, Geometric, and Harmonic Proportions] |
|
| Now it will be explained how the third term is extracted for each of these three proportions, when two of the terms are given: | ונבאר עתה איך יוצא הגבול השלישי לכל אחד מאלו השלשה אמצעיים כאשר היו שני חלקים מהם מונחים |
|
וזה שאם היו שני חלקים מאמצעי החשבון נלוים מונחים ונבקש הגבול השלישי |
|
הנה אנו נשימהו אם היה הדרוש הגבול הגדול מספר ששעור תוספתו על האמצעי כשעור תוספת האמצעי על הקטן |
|
ונמצא גם כן הגבול השלישי כאשר היה הגדול כאשר נכפול האמצעי עוד נשליך ממנו הגבול הקטן ומה שישאר הוא הגבול הגדול |
|
הנה כבר נאמר שאמצעי החשבון הוא אשר יוסיפו גבוליו קצתם על קצת בשוה ואינם מתיחסים הגבולים |
|
ואמרנו שכפל האמצעי מגבוליו כמו שני קצוותיו יחד |
|
וכדמיון זה נוציא הגבול הקטן אם היה הדרוש וזה שאנו נשים הגבול הקטן מספר יהיה חסרונו מהאמצעי כשעור חסרון האמצעי מהגדול |
|
או כפל האמצעי הנה נשליך ממנו הגבול הגדול ומה שישאר הוא הגבול הקטן |
|
אמנם אם היה הדרוש באמצעי |
|
אנו נקח חצי שני הקצוות מקובצים והוא האמצעי |
|
ואמנם איך יוצא הגבול השלישי אל אמצעי המדות |
|
הנה אם היה דרושנו הגבול הגדול |
|
אנו נשימהו מספר יחס האמצעי אליו כיחס הקטן אל האמצעי |
|
או נכה האמצעי בעצמו ומה שיצא נחלקהו על הגבול הקטן ומה שיצא בחלוק הוא הגבול הגדול |
|
וכבר בארנו לפנים שאמצעי המדות הוא אשר גבוליו מתיחסים ואין תוספתם שוה |
|
ובארנו הכאת שהאמצעי בעצמו כמו שני הקצוות מוכה אחד מהם באחר |
|
כי המספר אשר יצא מחלוקת אותו המספר על אחד משני המספרים המוכה אחד מהם באחר שוה לאשר לא יחלק עליו מהם |
|
ואם היה הדרוש הגבול האמצעי |
|
הנה אנו נקח שורש המתקבץ מהכאת אחד משני המספרים באחר ונשימהו האמצעי |
|
ואמנם איך נוציא הגבול השלישי מאמצעי החבור |
|
הנה אין אחד משני גבולי הקצוות אפשרי להוציאו |
|
וזה שיצטרך בהוצאת כל אחד מהם אל שתי ידיעות מוסכלות מארבעה מתיחסים חלוקים ביחס |
|
אחד מהם בגבול המוסכל עצמו |
|
והשני מותר הגבול המוסכל על הגבול האמצעי אם היה הגבול המוסכל הוא הגבול הגדול |
|
או מותר האמצעי על הקטן אם היה המוסכל הוא הקטן |
|
ואמנם הוצאת האמצעי |
|
הנה כבר נודע שהוא כאשר לוקח מותר הגדול על הקטן וחולק בשני חלקים יחס א' מהם אל האחר יחס הגדול אל הקטון |
|
כי הקטון משני חלקי המותר כאשר נוסף על הקטן משני הקצוות היה המתקבץ הוא האמצעי וכאשר חוסר החלק הגדול משני חלקי המותר מהגבול הגדול היה גם כן הוא האמצעי |
|
ואמנם אופן אחר ההכאה בם אנו נכה אחד משני הקצוות באחר ועוד נכפל מה שיתקבץ ונחלק מה שיתקבץ על שני הקצוות מקובצים ומה שיצא מהחלוקה הוא האמצעי |
| This is enough for the description of the three proportions that are recognized by the ancients. | וזה מספיק בתאר האמצעים השלשה המפורסמים אצל הקדמונים |
| The discussion on the remaining will be brief, as the ancients rarely used them. | ואמנם הנשארים נקצר המאמר עליהם למעוט השתמש הקדמונים מהם |
The Fourth Proportion |
האמצעי הרביעי |
|
הוא הראשון מהשבעה האמצעים הנשארים |
|
והוא אשר יאמר לו מקביל האמצעי החבוריי |
| When there are three terms, the ratio of the excess of the mean over the smaller to the excess of the greater over the mean is as the ratio of the greater to the smaller.
|
והוא שיהיו שלשה גבולים יחס מותר האמצעי על הקטן אל מותר הגדול על האמצעי כיחס הגדול אל הקטן |
|
כמו ג' ה' ו' |
|
וסגולת זה האמצעי הוא שהכאת הגדול באמצעי כפל הקטן באמצעי |
The Fifth Proportion |
והאמצעי החמשי |
|
והוא אחד משני המקבילים לאמצעי המדותיי |
| When there are three terms, the ratio of the excess of the greater over the mean to the excess of the mean over the smaller is as the ratio of the smaller to the mean.
|
הוא כאשר יהיו שלשה גבולים יחס מותר הגדול על האמצעי אל מותר האמצעי על הקטן כיחס הקטן אל האמצעי |
|
כמו ב' ד' ה' |
|
וסגלת זה שמה שיתקבץ מהכאת הגדול באמצעי כפל המתקבץ מהכאת הגדול בקטן |
|
ויתחייב מזה שיהיה לעולם יחס האמצעי אל הקטן הוא יחס הכפל |
The Sixth Proportion |
ואמנם האמצעי הששי |
|
והוא השני לשני המקבילים לאמצעי המדותי |
| When there are three terms, the ratio of the excess of the mean over the smaller to the excess of the greater over the mean is as the ratio of the greater to the mean.
|
הנה הוא כאשר היו שלשה גבולים יחס מותר האמצעי על הקטן אל מותר הגדול אל האמצעי כיחס הגדול אל האמצעי |
|
כמו א' ד' ו' |
|
וסגלת זה שמה שיתקבץ מהכאת המותר הגדול בגבול האמצעי שוה למה שיתקבץ מהכאת המותר הקטן בגבול הגדול |
| The proportions that were used from after Pythagoras until Plato's time are the three first proportions. | הנה האמצעים הנעשים מאחר פיתאגוריש עד זמן אפלאטון הם השלשה הראשונים |
| The other three are their opposites. | ואלו השלשה האחרים הם מקבילים |
| The four remaining were mentioned by the contemporaries and found to a small extent in the writings of the ancients. | ואמנם הארבעה הנשארים אשר זכרום החדשים ומעט מה שימצאו בספרי הקדמונים |
| Since they were mentioned literally, it is necessary to mention them - lest the author will be considered ignorant concerning them. | וכאשר נזכרו זכר פשוט כבר יחוייב שנזכירם כדי שלא יחשוב חושב שאנו עזבנום להכלותנו בם והם אלו |
The Seventh Proportion |
האמצעי השביעי |
| When there are three terms, the ratio of the excess of the greater over the smaller to the excess of the mean over the smaller is as the ratio of the greater to the smaller.
|
הוא כאשר היו שלשה גבולים יחס מותר הגדול על הקטן אל מותר האמצעי על הקטן כיחס הגדול אל הקטן |
|
כמו ו' ח' ט' |
The Eighth Proportion |
והאמצעי השמיני |
| When the setting of the terms is such that the ratio of the greater to the smaller is as the ratio of the excess of the greater over the smaller to the excess of the greater over the mean.
|
הנה הוא כאשר היתה הנחת הגבולים כן יחס הגדול אל הקטן כיחס מותר הגדול על הקטן אל מותר הגדול על האמצעי |
|
כמו ו' ז' ט' |
The Ninth Proportion |
והאמצעי התשיעי |
| When there are three terms, the ratio of the excess of the greater over the smaller to the excess of the mean over the smaller is as the ratio of the mean to the smaller.
|
הוא כאשר היו שלשה גבולים יחס מותר הגדול על הקטן אל מותר האמצעי על הקטן כיחס האמצעי אל הקטן |
|
כמו ד' ו' ז' |
The Tenth Proportion |
אמנם האמצעי הי |
| When there are three terms, the ratio of the excess of the greater over the smaller to the excess of the greater over the mean is as the ratio of the mean to the smaller.
|
כאשר היו ג' גבולים יחס מותר הגדול על הקטן אצל מותר הגדול על האמצעי כיחס האמצעי אל הקטן |
|
כמו ג' ה' ח' |
| We have completed all of them in this diagram: | והנה השלמנו כלם בזאת הצורה |
| its property: equality of the difference and equality of the sum of the two extremes to double the mean | 3 | 2 | 1 | arithmetic proportion |
| its property: equality of the ratio and the ratio of the mean to the smaller is as the ratio of the difference between the two greater to the difference between the two smaller | 4 | 2 | 1 | geometric proportion |
| its property: the ratio of the greater to the smaller is as the ratio of the difference between the two greater to the difference between the two smaller | 6 | 4 | 3 | harmonic proportion |
| its property: the ratio of the greater to the smaller is as the ratio of the difference between the two smaller to the difference between the two greater | 6 | 5 | 3 | opposite to the harmonic proportion |
| its property: the ratio of the mean to the smaller is as the ratio of the difference between the two smaller to the difference between the two greater | 5 | 4 | 2 | opposite to the geometric proportion |
| its property: the ratio of the greater to the mean is as the ratio of the difference between the two smaller to the difference between the two greater | 6 | 4 | 1 | opposite to the geometric proportion |
| its property: the ratio of the excess of the greater over the smaller to the excess of the mean over the smaller is as the ratio of the greater to the smaller | 9 | 8 | 6 | seventh proportion |
| its property: the ratio of the greater to the smaller is as the ratio of the excess of the greater over the smaller to the excess of the greater over the mean | 9 | 7 | 6 | eighth proportion |
| its property: the ratio of the excess of the greater over the smaller to the excess of the mean over the smaller is as the ratio of the mean to the smaller | 7 | 6 | 4 | ninth proportion |
| its property: the ratio of the excess of the greater over the smaller to the excess of the greater over the mean is as the ratio of the mean to the smaller | 8 | 5 | 3 | tenth proportion |
| סגולתו שווי היתרון ושווי שני הקצוות מקובצים לאמצעי כפול | ג | ב | א | האמצעי החשבוני |
| סגולתו שווי היחס ושיחס האמצעי לקטן כיחס מותר מה שבין שני הגדולים אל המותר שבין הקטנים | ד | ב | א | האמצעי המדותי |
| סגולתו שיחס הגדול אל הקטן כיחס מותר מה שבין שני הגדולים אל המותר שבין שני הקטנים | ו | ד | ג | האמצעי החבוריי |
| סגולתו שיחס הגדול לקטן כיחס מותר מה שבין שני הקטנים אל המותר שבין שני הגדולים | ו | ה | ג | המקביל לחבוריי |
| סגולתו שיחס האמצעי אל הקטן כיחס מותר מה שבין שני הקטנים אל מותר שבין שני הגדולים | ה | ד | ב | המקביל למדותיי |
| סגולתו שיחס הגדול לאמצעי כיחס המותר שבין שני הקטנים אל מותר שבין שני הגדולים | ו | ד | א | המקביל ג"כ למדותיי |
| סגולתו שיחס מותר הגדול על הקטן אל מותר האמצעי על הקטן כיחס הגדול אל הקטן | ט | ח | ו | האמצעי השביעי |
| סגולתו שיחס הגדול לקטן כיחס מותר הגדול על הקטן אל מותר הגדול על האמצעי | ט | ז | ו | האמצעי השמיני |
| סגולתו שיחס מותר הגדול על הקטן אל מותר האמצעי על הקטן כיחס האמצעי אל הקטן | ז | ו | ד | האמצעי התשיעי |
| סגולתו שיחס מותר הגדול על הקטן אל מותר הגדול על האמצעי כיחס האמצעי אל הקטן | ח | ה | ג | האמצעי העשירי |
The Perfect Proportion that Encompasses the body |
הדבור באמצעי השלם המקיף במוגשם | ||||||||
|---|---|---|---|---|---|---|---|---|---|
| The proportion that has three terms, as the span of its ends is composed of two intervals, which are: | האמצעי בעל הג' גבולים מפני שהקפת תכליותיו מורכבת מב' המרחקים והם | ||||||||
|
מרחק מה שבין הגדול והאמצעי | ||||||||
|
ומרחק מה שבין אמצעי והקטן | ||||||||
| As in the surface that is composed of length and breadth. | כמו אותם אל הפשוט אשר הוא מורכב מהארך והרחב | ||||||||
| The proportions that are imagined in the solid body are more perfect proportions, and it is impossible that there would be a proportion that deserves to be named perfect more than them, as the perfection of the solid body is complete, since it is impossible by nature that it will get more than three dimensions, which are the length, the breadth, and the depth. | ואמנם האמצעים המדומים בגשם הנה הם יותר שלמים האמצעיים וא"א להיות אמצעי יותר ראוי בשם השלימות מהם כמו שהמוגשם כבר יכלה שלמותו אחר שאי אפשר בטבע שיקבל תוספת על המרחקים הג' אשר הם הארך והרחב והעומק | ||||||||
| Therefore, the proportion that has four terms, as the span of its ends is composed of three intervals, which are: | הנה אם כן האמצעי בעל הד' גבולים מפני שההקפה אשר לתכליותיו היא מורכבת מג' מרחקים והם | ||||||||
|
מרחק מה שבין הראשון והב' | ||||||||
|
ומרחק מה שבין הב' לשלישי | ||||||||
|
ומרחק מה שבין הג' לד' | ||||||||
| It is by reason and necessary the perfect proportion imagined in the body. | היה בדין ובראוי הוא האמצעי השלם הדומה במוגשם | ||||||||
| It has this analogy by reason, since the three dimensions of the body are in four limits, which are the unit, the side, the surface, and the body. | ובדין לו זה הדמיון כי היו מרחקי הגשם הג' אמנם עמדו מד' גבולים והם האחדות והצלע והפשוט והגשם | ||||||||
| For the space between the unit and the body is the body itself and it consists of the three intervals, which are: the intervals between the unit and the side, the intervals between the side and the surface, and the intervals between the surface and the body. | כי מרחק מה שבין האחדות והגשם הוא המוגשם עצמו והוא מורכב מהג' רחקים אשר הם מרחק מה שבין האחדות והצלע ומרחק מה שבין הצלע והפשוט ומרחק מה שבין הפשוט והגשם | ||||||||
| Also the perfect proportion that has four limits is the span of its ends, and one finds that this span consists of the three intervals, which are: the interval from the first term to the second, the interval from the second to the third, and the interval from the third to the fourth. | וכן האמצעי השלם בעל הד' גבולים הוא הקפת תכליותיו ותמצא אותה ההקפה מורכבת מג' המרחקים אשר הם מרחק הגבול הראשון מהב' ומרחק הב' מהג' והמרחק הג' מהד' | ||||||||
| Nicomachus brought an example for this perfect solid comprehensive proportion after demonstrating that the comprehension is by the properties of the three proportions, i.e. the arithmetic proportion, the geometric proportion, and the harmonic proportion. | וכבר הניח ניקומכוש לזה האמצעי השלם המוגשם המקיף דמיון אחר הראנו כי ההקפה בסגולות האמצעיים הג' ר"ל אמצע החשבון ואמצע המדות אמצע החבור | ||||||||
| This is the diagram of the example that Nicomachus brought: | וזה צורתו המשל שהביא ניקומאכוש | ||||||||
|
| ||||||||
|
ואמנם סגולת האמצעי החשבון הנמצאת בזה האמצעי השלם המוגשם המקיף הנה יראה בג' גבולי' מהם והם הראשון והג' והד' וזה לפי שמותר הרביעי על הג' הוא מותר הג' על הראשון ושהראשון והד' מקובצים כמוכפל האמצעי והיא הסגולה שזכרנו וכאשר היה זה כן הנה כבר יתחייבו לו כל הסגולות אשר קדם זכרם לאמצע החשבון | ||||||||
|
ואמנם סגולת אמצע המדות הנמצאת בזה האמצעי השלם המוגשם המקיף תראה בגבולי' הד' יחד כאשר יוחס הראשון אל הג' והב' אל הד' וזה לפי שהיחס יהיה אז אחד ויהיה מוכה ב' הקצוות א' מהם באחר כמוכה ב' האמצעיי' א' מהם באחר וכאשר היה זה כן יתחייבו לו שאר הסגולות הנמצאות לאמצעי המדות | ||||||||
|
ואמנם סגולת האמצעי החבור אשר תמצא בזה האמצעי השלם המוגשם המקיף תראה בג' מגבוליו כמו שנראה באמצעי החשבון והראות זה יהיה כאשר ייוחס הד' אל הב' והב' אל הראשון כי יהיה אז יחס מותר הד' על הב' אל הד' כיחס מותר הב' על הראשון וכאשר היה זה כן הנה יתחייבו לו ג"כ שאר הסגולות הנמצאו' אל אמצעי החבור | ||||||||
| The properties of the arithmetic proportion are evident in three terms, likewise the properties of the harmonic proportion are in three terms, however the evidence of the properties of the geometric proportion is different, as they are evident when four terms are applied together, since the virtue of these is mean between the two other techniques and it takes the appropriate from both together, thus what is different in them is summed by it. | ואמנם נראו סגולות אמצעי החשבון בג' גבולים וסגולות אמצעי החבור בג' גבולים וחלוף זה הראות סגולות אמצעי המדות כאשר השתמש בהראותם ד' גבולים יחד לפי שהמדה באלו היא אמצעית בין ב' המלאכות האחרות ותקח הראוי מהן יחד והנה יתקבץ בה מה שתפריש בם | ||||||||
| Apart than that, we say that all the harmonies that exist in the art of music are evident in this perfect solid comprehensive proportion: | וזולת זה אנו נאמר כי כבר יראה בזה האמצעי השלם המוגשם המקיף כל ההסכמות הנמצאות במלאכת החבור | ||||||||
|
אם ההסכמה בד' והיא על יחס דמיון ושליש תראה מהקשת הראשון אל הב' ותרא' ג"כ מהקשת הג' אל הד' | ||||||||
|
ואמנם ההסכמה בחומש והוא על יחס דמיון וחצי תרא' מהקשת הראשון אל הג' ותראה גם כן מהקשת השני אל הרביעי | ||||||||
|
ואמנם ההסכמה בכל והוא על יחס הכפל השניי תראה מהקשת הראשון אל הד' | ||||||||
|
ואמנם הזמן והוא על יחס דמיון ושמינית תראה מהקשת מה שבין הב' והג' | ||||||||
|
וזה הזמן הוא שעור משותף לכל הליחנים והוא הפחות שבמרחקים המקבילים בליחנים להחלק | ||||||||
|
והוא היתרון אשר בין ההסכמה בחומש וההסכמה בד' כמו שהשמינית הוא המותר בין המוסיף חצי ובין המוסיף שליש | ||||||||
| I have concluded showing these harmonies in this perfect solid comprehensive proportion in this diagram. | וכבר השלמתי לך איך יראו אלו ההסכמות מזה האמצעי השלם המקיף המוגשם בצורה זו | ||||||||
| Over and done. | תם ונשלם | ||||||||
| Praise to God, the Creator of the world. | שבח לאל בורא עולם | ||||||||
Appendix: Bibliography
Nicomachus of Gerasa
2nd century C. E
’Αριθμητικής είσαγωγής βιβλία β – Introduction to Arithmetic
Critical Edition:
- Nicomachus of Gerasa. Introduction to Arithmetic. trans. Martin L. D'Ooge. Chicago IL: Encyclopaedia Britannica, 1955.[Great Books of the Western World, vol.11], pp. 811 – 848.
– Hebrew translation –
Qalonymos ben Qalonymos (known as Maestro Calo or Callus)
South of France, 1286/7-after 1329
Sefer ha-Aritmitiqa / Aritmaiti
1317
Manuscripts:
- 1) Halle, Universitätsbibliothek Yb Qu. 5/1 (IMHM: f 71790), ff. 1r-54r (15th century)
- 2) München, Bayerische Staatsbibliothek, Cod. hebr. 36/14 (IMHM: f 1166), ff. 144r-164r (Istanbul, 1485)
- 3) New York, Jewish Theological Seminary Ms. 2449/2 (IMHM: f 28702), ff. 107r-153r (15th century)
- [JTS2449]
- 4) Paris, Bibliothèque Mazarine 4478/1 (IMHM: f 4414), ff. 194-296 (15th century)
- 5) Paris, Bibliothèque Nationale de France heb. 1028/1 (IMHM: f 15720), ff. 1r-54r (1342)
- 6) Paris, Bibliothèque Nationale de France heb. 1029/1 (IMHM: f 15721), ff. 1r-30v (15th – 16th century)
- 7) Paris, Bibliothèque Nationale de France heb. 1093/2 (IMHM: f 15043), ff. 127r-155v (15th century)
- 8) Paris, Bibliothèque Nationale de France heb. 1095/8 (IMHM: f 15045), ff. 177-226 (15th century)
The transcript is based mainly on manuscript Halle
Bibliography:
- Freudenthal, Gad et Lévy Tony. 2004. De Gérase à Bagdad: Ibn Bahriz, al-Kindi, et leur recension arabe de l’Introduction arithmétique de Nicomaque, d’après la version hébraïque de Qalonymos ben Qalonymos d’Arles, In R. Morelon et A. Hasnawi eds. De Zénon d’Elée à Poincaré. Recueil d’études en hommage à Roshdi Rashed. Louvain-Paris. pp. 479-544.
- Freudenthal, Gad and Mauro Zonta. 2007. Remnants of Habīb ibn Bahrīz’s Arabic translation of Nicomachus of Gerasa’s Introdaction to Arithmetic, in Y. Tzvi Langermann and Josef Stern eds. Adaptations and Innovations: Studies on the interaction between Jewish and Islamic thought and literature from the early Middle Ages to the late twentieth century, dedicated to Professor Joel L. Kraemer. Paris, Louvain and Dudley: Peeters, 2007. pp.67-82.
- ———. 2009. Nicomachus of Gerasa in Spain, Circa 1100: Abraham bar Ḥiyya’s Testimony, Aleph 9.2 (2009), pp. 189-224.
- Langermann, Y. Tzvi. 2001. Studies in Medieval Hebrew Pythagoreanism: Translations and Notes to Nicomachus; Arithmological Texts, Micrologus IX, pp. 219–36.
- Sarfatti, Gad ben ‛Ami. 1968. Mathematical Terminology in Hebrew Scientific Literature of the Middle Ages. Jerusalem: Magnes Press, pp. 200-214.
- Steinschneider, Moritz. 1894. Miscellen 26. Nikomachus, Arithmetik, Monatsschrift für die Geschichte und Wissenschaft des Judenthums 38, pp. 68–77.
– Summary –
Manuscript:
- Oxford, Bodleian Library MS Mich. 29/3 (IMHM: f 20994), ff. 131v-158r (cat. Neub. 2302, 3; 18th century)
– Commentaries on the Introduction to Arithmetic by Nicomachus –
Caleb Afendopolo
Constantinople, 1499
Manuscript:
- Berlin, Staatsbibliothek (Preußischer Kulturbesitz) Or. Qu. 760 (IMHM: f 1742) (1499)
Bibliography:
- Steinschneider, Moritz. 1896. Miscellen 36. Kaleb Afednopolo's encyklopädische Einteilung der Wissenschaften, Monatsschrift für die Geschichte und Wissenschaft des Judenthums 40, pp. 90–94.
A certain Yosef
Manuscript:
- Moscow, Russian State Library, Ms. Guenzburg 340/1 (IMHM: f 47750), ff. 2r-18v (16th century)
Abraham Yerushalmi
Manuscripts:
- Moscow, Russian State Library, Ms. Guenzburg 340/2 (IMHM: f 47750), ff. 19r-23r (16th century)
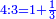 ]
]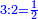 ]
]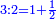 ]
] ]
]![\scriptstyle n=\frac{1}{2}\sdot\left[\left(n+1\right)+\left(n-1\right)\right]](/mediawiki/images/math/0/8/a/08a331a4b14c89dd6b9cea191a31ef06.png)
![\scriptstyle n=\frac{1}{2}\sdot\left[\left(n+2\right)+\left(n-2\right)\right]](/mediawiki/images/math/3/8/0/380f7d2cc486e55ce5fc116c2f382b67.png)
![\scriptstyle n=\frac{1}{2}\sdot\left[\left(n+m\right)+\left(n-m\right)\right]](/mediawiki/images/math/d/1/f/d1f23c2ce19c992ceead63ae37670955.png)
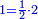

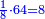 → 8 is even-times-even
→ 8 is even-times-even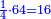 → 4 and 16 are even-times-even
→ 4 and 16 are even-times-even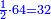 → 2 and 32 are even-times-even
→ 2 and 32 are even-times-even which is derived from 9
which is derived from 9 which is derived from 3
which is derived from 3


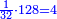
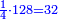
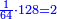
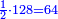

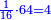
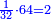



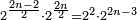

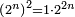
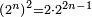
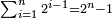
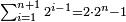
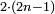

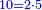
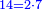
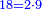
 ], as illustrated in their terms.
], as illustrated in their terms.
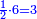
 , and whose half is 4:
, and whose half is 4: 
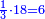
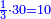
![\scriptstyle\left[2\sdot\left(2n+1\right)\right]+\left[2\sdot\left[\left[2\sdot\left(n+1\right)\right]+1\right]\right]=\left[2\sdot\left(2+1\right)\right]+\left[2\sdot\left[\left(2\sdot2n\right)+1\right]\right]](/mediawiki/images/math/0/3/b/03b1aebe88db1e369e31ef8013f61229.png)
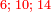 ] When setting 6 as the smaller extreme of the even-times-odd numbers and 14 as the greater extreme, their sum is 20, and the mean, which is 10, when it is doubled is the same.
] When setting 6 as the smaller extreme of the even-times-odd numbers and 14 as the greater extreme, their sum is 20, and the mean, which is 10, when it is doubled is the same.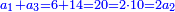
 ] When setting 18 as the greater extreme, the sum of the two extremes is 24, and the two means, which are 10 and 14, are also 24.
] When setting 18 as the greater extreme, the sum of the two extremes is 24, and the two means, which are 10 and 14, are also 24.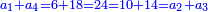
 ] When setting 4 as the smaller extreme of [the even-times-even numbers] and 16 as the greater extreme, the product of 4 by 16 is equal to the product of the mean, which is 8, by itself, which is 64.
] When setting 4 as the smaller extreme of [the even-times-even numbers] and 16 as the greater extreme, the product of 4 by 16 is equal to the product of the mean, which is 8, by itself, which is 64.
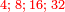 ] When setting 32 as the greater extreme, the product of 4 by 32 is 128 and it is equal to the product of the two means one by the other, which are 8 and 16.
] When setting 32 as the greater extreme, the product of 4 by 32 is 128 and it is equal to the product of the two means one by the other, which are 8 and 16.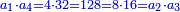
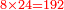 ] is far from 24 by two intermediary terms [breadthwise], yet it is not far by two intermediary terms from the product [of the first term of the even-times-even numbers by the first term in the second column of the even-times-even-times-odd numbers], but from the multiplied [i.e. by the first term in the second column of the even-times-even-times-odd numbers] itself.
] is far from 24 by two intermediary terms [breadthwise], yet it is not far by two intermediary terms from the product [of the first term of the even-times-even numbers by the first term in the second column of the even-times-even-times-odd numbers], but from the multiplied [i.e. by the first term in the second column of the even-times-even-times-odd numbers] itself. → from 7
→ from 7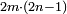 .
. .
. , so that the multiplied odd number divides it by the number of times by which it is multiplied, and [the product] is also divided by the number which multiplies the prime incomposite odd number, that is by the multiplied odd number.
, so that the multiplied odd number divides it by the number of times by which it is multiplied, and [the product] is also divided by the number which multiplies the prime incomposite odd number, that is by the multiplied odd number. .
.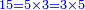 .
.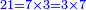 .
.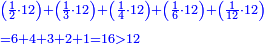

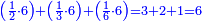
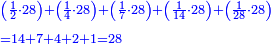
 is not a simple ratio.
is not a simple ratio. → this is the double sesquialter ratio, which is not a simple ratio.
→ this is the double sesquialter ratio, which is not a simple ratio.
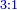
 and
and 
 ;
;  ;
;  ; and so on until the end of the numbers that were set.
; and so on until the end of the numbers that were set.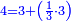
 ]: Starting by arranging the numbers by natural order, from one to ten, in the first row.
]: Starting by arranging the numbers by natural order, from one to ten, in the first row. ]: The double ratio in the second row, adjacent to the first row.
]: The double ratio in the second row, adjacent to the first row. ]: The triple ratio in the third row.
]: The triple ratio in the third row. ]: The quadruple ratio in the fourth row.
]: The quadruple ratio in the fourth row.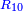 ]
] ] that meet on the square 4 and extend to the surface 1-100
] that meet on the square 4 and extend to the surface 1-100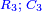 ] that meet on the square 9 and extend to the surface 1-100
] that meet on the square 9 and extend to the surface 1-100 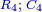 ] by four.
] by four.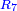 ] to its precedecessor 36 on this row is as the ratio of 7 on the first row [
] to its precedecessor 36 on this row is as the ratio of 7 on the first row [ ]
] ] to its preceding 6 on this column is as the ratio of 42 on the seventh column [
] to its preceding 6 on this column is as the ratio of 42 on the seventh column [ ] to its predecessor 36 on the same column [
] to its predecessor 36 on the same column [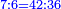 ]
] ] and the row that goes from 6 on the first column [
] and the row that goes from 6 on the first column [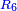 ] meet on the square 36, and 36 is the term related to each of the terms 42 on both cross-lines, as both lines intersect on the square 36.
] meet on the square 36, and 36 is the term related to each of the terms 42 on both cross-lines, as both lines intersect on the square 36.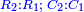 ]
] ]
] ]
]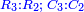 ]
]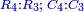 ]
]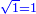
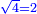
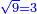
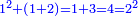
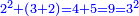
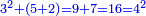



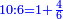 ]
] ]
] ]
] ]
]
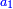 ]
] ]
] ]
]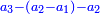 ]
]
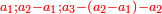 ]
] sesquialter ratio
sesquialter ratio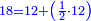
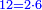
 triple ratio
triple ratio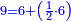


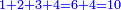


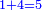
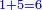
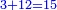
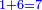
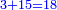
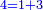
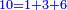
 , when four units are composed with them
, when four units are composed with them  , the sum is eight, eight is two by two by two
, the sum is eight, eight is two by two by two  , and this is equal in the three dimension, i.e. the length, breadth and depth.
, and this is equal in the three dimension, i.e. the length, breadth and depth.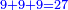 , which is the product of three by three by three
, which is the product of three by three by three 
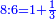
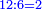

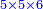
 the five returns, expressed in the total.
the five returns, expressed in the total.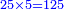 the five is again expressed in the total.
the five is again expressed in the total.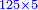


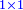








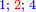
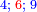

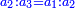 .
.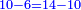

 , and this and its like were already explained in the first section.
, and this and its like were already explained in the first section.
 ], but the excess of 4 over 2 is two [
], but the excess of 4 over 2 is two [ ] - the ratio is one and the same, while the additions are different.
] - the ratio is one and the same, while the additions are different.  ], but the ratio differs, as the ratio of 6 to 4 is not the ratio of 4 to 2 [
], but the ratio differs, as the ratio of 6 to 4 is not the ratio of 4 to 2 [ ].
].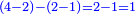 ], and so on.
], and so on.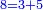 .
. .
.
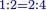 ]
] ].
].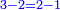 ]; and vice versa.
]; and vice versa. ].
]. ]; and vice versa.
]; and vice versa.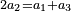
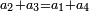
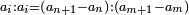
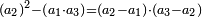

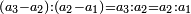
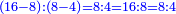
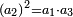
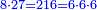
 , 48 is not a cube and not a rooted surface.
, 48 is not a cube and not a rooted surface.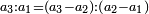
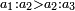 ]
]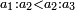 ]
]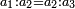 ]
]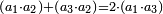
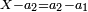
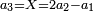
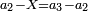


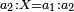
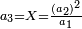
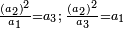 ]
]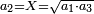
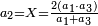
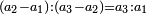
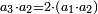 is incorrect]
is incorrect]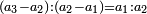
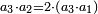 is incorrect]
is incorrect]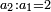 is also incorrect]
is also incorrect]
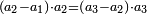
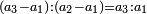
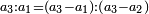
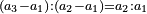
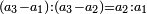
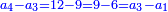 ]
]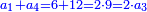 ]
]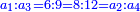 ]
]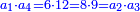 ]
] ]
] ]
]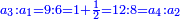 ]
]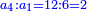 ]
]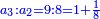 ]
]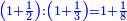 ]
]