Difference between revisions of "האנציקלופדיה של אבו אלצלת"
From mispar
(→[Sums]) |
(→Heteromecic and square numbers) |
||
| (411 intermediate revisions by the same user not shown) | |||
| Line 1: | Line 1: | ||
| + | {{#annotpage: author="Abū al-Ṣalt", translator="Don Benveniste b. Lavi", city="Saragossa", time="1395-1435", peshat_author="00000703", peshat_title="00002054"}} | ||
__TOC__ | __TOC__ | ||
<br> | <br> | ||
{| | {| | ||
|- | |- | ||
| − | | | + | |<big>The Book of Nicomachus on the Numerical Properties</big> |
| − | + | |style="text-align:right;"|<big>ספר ניקומאכוש בסגלות המספריות</big> | |
|- | |- | ||
| − | | | + | |Don Benveniste b. Lavi, may his soul rest in heaven, translated it from Arabic to the holy language in the year 195 of creation. |
|style="text-align:right;"|העתיקו בסרגוסא דארגו' השר דון בנבשת בן לביא נ"ע מלשון הגרי ללשון הקדש שנת קצ"ה ליצירה | |style="text-align:right;"|העתיקו בסרגוסא דארגו' השר דון בנבשת בן לביא נ"ע מלשון הגרי ללשון הקדש שנת קצ"ה ליצירה | ||
|- | |- | ||
| − | | | + | |In any place where A"B is written in the work, it means: Benveniste said. |
|style="text-align:right;"|ובכל מקום הכתוב בגיליון א"ב ר"ל אמר בן בנשתי‫' | |style="text-align:right;"|ובכל מקום הכתוב בגיליון א"ב ר"ל אמר בן בנשתי‫' | ||
|- | |- | ||
| Line 22: | Line 23: | ||
| | | | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | *Arithmetic is a theoretical science, its subject is the absolute number and its types, its purpose is the understanding of its concepts, of what follows from it, of its properties and its general essential accidents. |
| + | |style="text-align:right;"|{{#annot:term|1174,365|5v1l}}חכמת המספר{{#annotend:5v1l}} חכמה עיונית נושאה המספר המשולח אליו ומיניו והכונה בה העמידה על משיגיו ועל המתחייב אליו וסגלותיו ובכלל מקריו העצמיים | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | *The essence of the number means the number is a sum of units and its definition is that it is a divisible quantity that exists from units. |
| + | |style="text-align:right;"|ומהות המספר הנה תאמר המספר קבוץ אחדים וגדרו שהוא כמות מתחלק מתקיים מאחדים | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"|ויש לוקחין | + | *Some understand unities as units, since it indicates the nature of the number, as the sum of the units is not the number itself, whose intention here is its attribute and its definition and in general the announcement of its essence, but the counted that are the subjects of the number, as a sum of people, or horses, or others. |
| + | |style="text-align:right;"|ויש לוקחין האחדותיות תמורת האחדים לפי שהוא יותר מורה על טבע המספר, לפי שקבוץ האחדים אינו המספר עצמו המכוון הנה תאמו וגדרו ובכלל הודעת מהותו אבל הנספרים אשר הם נושאי המספר כקבוץ מהאנשים או מהסוסי' או זולת זה | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | :Whereas the sum of unities is the number itself that is summed from the duplication of the unity, by which it is said "one" about each thing of the counted. |
| + | |style="text-align:right;"|וקבוץ האחדותיות הוא המספר עצמו המקובץ מהכפלת האחדות אשר בה יאמ' לכל דבר מהנספרים אחד | ||
|- | |- | ||
| − | | | + | | |
| + | *The absolute number is divided first into two types: even number and odd number. | ||
|style="text-align:right;"|והמספר המשולח יתחלק ראשנה לשני מינים זוג ונפרד | |style="text-align:right;"|והמספר המשולח יתחלק ראשנה לשני מינים זוג ונפרד | ||
|- | |- | ||
| − | |{{#annot:definition|63|4hko}} | + | | |
| − | |style="text-align:right;"| | + | :*{{#annot:definition|63|4hko}}The even number is any number that is divisible into two equal parts, such as 2 and 4. |
| + | |style="text-align:right;"|והזוג הוא כל מספר שיתחלק לשני חלקים שוים כשנים וארבע{{#annotend:4hko}} | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | :*{{#annot:definition|65|mnXP}}The odd number is any number that is indivisible into two equal parts. |
| + | |style="text-align:right;"|והנפרד הוא כל מספר שא"א שיחלק לשני חלקים שוים{{#annotend:mnXP}} | ||
|- | |- | ||
| − | |'' | + | |Our intention is to add the known way of arithmetic to the mathematical science that we have prefaced and what is customary to bring concerning it according to the way that was spread from the ''Book of Elements'' that presented many principles in arithmetic, and establishing this way by the realization of this principles. |
| − | |style="text-align:right;"|כונתינו שנחבר אל מה שהקדמנו מהחכמות הלמודיות האופן הידוע בארתימטיקי ומה שפשט המנהג להביאו בו כפי האופן אשר פשט על | + | |style="text-align:right;"|כונתינו שנחבר אל מה שהקדמנו מהחכמות הלמודיות האופן הידוע בארתימטיקי ומה שפשט המנהג להביאו בו כפי האופן אשר פשט על ספר היסודות כבר נתן שרשים רבים בחכמת המספר והנחת זה האופן אצל ההגעה באותם השרשים |
|- | |- | ||
| − | | | + | |Many of the geometric shapes that have relation with the multiplication, division and ratio, may be related to the number and the rules of number of this book may be established upon them. |
|style="text-align:right;"|וכבר אפשר שתתחברנה הרבה מהתמונות התשברתיות אשר להם התלות בהכאה והחלוק ובענייני היחס אל המספר ושתישבו ממנו משפטי המספר זה הספר והנה זה אליך | |style="text-align:right;"|וכבר אפשר שתתחברנה הרבה מהתמונות התשברתיות אשר להם התלות בהכאה והחלוק ובענייני היחס אל המספר ושתישבו ממנו משפטי המספר זה הספר והנה זה אליך | ||
|- | |- | ||
| − | | | + | |As for the essence of the number - some of it is already known in the ''Book of Categories'' and a hint about it was alluded in the ''Book of Elements'', also in metaphysics one reaches to truths about it. |
| − | |style="text-align:right;"|אמנם מהות המספר הנה כבר ידעת | + | |style="text-align:right;"|אמנם מהות המספר הנה כבר ידעת בספר קאטיגוריאש ממנו דבר מה ונרמז לך בספר היסודות אליו רמז עוד יבא אליך בחכמה העליונה ממנו אמתות |
| + | |- | ||
| + | |Likewise for its two parts, which are the even number and the odd number. | ||
| + | |style="text-align:right;"|וכמו כן הענין בשני חלקיו אשר הם הזוג והנפרד | ||
| + | |- | ||
| + | |You already know the absolute prime, the absolute composite, and the relative composite from the ''Book of Element''. | ||
| + | |style="text-align:right;"|וכבר ידעת מספר היסודות הראשון והמורכב מוחלטים והמורכב בצרוף | ||
| + | |- | ||
| + | |You know the even and the odd, the even-times-odd, the even-times-even, and the even-times-even-times-odd, as well as the deficient number, the perfect number and the superabundant number. | ||
| + | |style="text-align:right;"|וידעת הזוג והנפרד וזוג הנפרד וזוג הזוג וזוג הזוג והנפרד וידעת המספר החסר והשלם והנוסף | ||
| + | |- | ||
| + | |We are not obligated to simplify [?] the mentioning of these matters for you, but we will endeavor to present the properties to you. | ||
| + | |style="text-align:right;"|ואיננו מחוייב אלינו הפשת זכרון אלה העניינים לך אבל שנשתדל להביא הסגלות אליך | ||
| + | |} | ||
| + | {| | ||
|- | |- | ||
| | | | ||
=== Properties of the Absolute Number === | === Properties of the Absolute Number === | ||
| − | + | | | |
| − | |style="text-align:right;"|ונזכור <big> | + | |- |
| + | |Mentioning the properties of the absolute number: | ||
| + | |style="text-align:right;"|ונזכור <big>סגלות המספר המשולח</big> | ||
|- | |- | ||
| | | | ||
| − | * | + | *The first of them and the most famous: every number is half [the sum of] its two sides, which are the numbers that are next to it at the same distance from the side of the subtraction and the addition: <math>\scriptstyle n=\frac{1}{2}\sdot\left[\left(n-m\right)+\left(n+m\right)\right]</math> |
| − | |style="text-align:right;"|והנה ראשנה שבהם והיותר מפורסמת | + | |style="text-align:right;"|והנה ראשנה שבהם והיותר מפורסמת שכל מספר הנה הוא חצי שתי פאותיו והם שני המספרים הנלוים אליו מצד המעוט והרבוי במרחק שוה |
|- | |- | ||
| | | | ||
| − | : | + | ::For example: <math>\scriptstyle{\color{blue}{\frac{1}{2}\sdot\left(6+4\right)=\frac{1}{2}\sdot\left(7+3\right)=\frac{1}{2}\sdot\left(8+2\right)=\frac{1}{2}\sdot\left(9+1\right)}}</math> |
|style="text-align:right;"|המשל בזה החמשה הנה הוא חצי ששה וארבעה וחצי שבעה ושלשה וחצי שמנה ושנים וחצי תשעה ואחד | |style="text-align:right;"|המשל בזה החמשה הנה הוא חצי ששה וארבעה וחצי שבעה ושלשה וחצי שמנה ושנים וחצי תשעה ואחד | ||
|- | |- | ||
| | | | ||
| − | * <math>\scriptstyle2n=\left(n-m\right)+\left(n+m\right)</math> | + | *Its double is equal to [the sum of] its two sides <math>\scriptstyle2n=\left(n-m\right)+\left(n+m\right)</math> |
|style="text-align:right;"|והנה יהיה כפלו שוה לשתי פאותיו | |style="text-align:right;"|והנה יהיה כפלו שוה לשתי פאותיו | ||
|- | |- | ||
| | | | ||
| − | * <math>\scriptstyle\frac{1}{2}\sdot n=\frac{1}{4}\sdot\left[\left(n-m\right)+\left(n+m\right)\right]</math> | + | *Its half [is equal to] a quarter of [the sum of] its two sides <math>\scriptstyle\frac{1}{2}\sdot n=\frac{1}{4}\sdot\left[\left(n-m\right)+\left(n+m\right)\right]</math> |
|style="text-align:right;"|וחציו לרביעית שתי פאותיו | |style="text-align:right;"|וחציו לרביעית שתי פאותיו | ||
|- | |- | ||
| | | | ||
| − | * <math>\scriptstyle n^2=\left[\left(n-1\right)\sdot\left(n+1\right)\right]+1</math> | + | *For every number, its square is equal to the product of its two close sides, one of them by the other, plus one <math>\scriptstyle n^2=\left[\left(n-1\right)\sdot\left(n+1\right)\right]+1</math> |
|style="text-align:right;"|וכל מספר יהיה מרובעו שוה להכאת שתי פאותיו הקרובות אחת מהם באחרת עם תוספת אחד | |style="text-align:right;"|וכל מספר יהיה מרובעו שוה להכאת שתי פאותיו הקרובות אחת מהם באחרת עם תוספת אחד | ||
|- | |- | ||
| | | | ||
| − | : | + | ::Such as: |
| + | ::*<math>\scriptstyle{\color{blue}{2^2=\left(1\sdot3\right)+1}}</math> | ||
|style="text-align:right;"|כמו מרובע שנים אשר הוא מהכאת שלשה באחד ותוספת אחד | |style="text-align:right;"|כמו מרובע שנים אשר הוא מהכאת שלשה באחד ותוספת אחד | ||
|- | |- | ||
| | | | ||
| − | : | + | ::*<math>\scriptstyle{\color{blue}{3^2=\left(2\sdot4\right)+1}}</math> |
|style="text-align:right;"|וכמו מרובע שלשה אשר הוא מהכאת ארבעה בשנים ותוספת אחד | |style="text-align:right;"|וכמו מרובע שלשה אשר הוא מהכאת ארבעה בשנים ותוספת אחד | ||
|- | |- | ||
| | | | ||
| − | : | + | ::*<math>\scriptstyle{\color{blue}{4^2=\left(3\sdot5\right)+1}}</math> |
|style="text-align:right;"|וכמו מרובע ארבעה אשר הוא מהכאת ג' בחמשה ותוספת אחד | |style="text-align:right;"|וכמו מרובע ארבעה אשר הוא מהכאת ג' בחמשה ותוספת אחד | ||
|- | |- | ||
| | | | ||
| − | * <math>\scriptstyle n^2 | + | *Also, for every number, its square exceeds the product of its two sides that are at the same distance, whichever they are, one of them by the other, by the square of the number of the terms between them <math>\scriptstyle n^2-\left[\left(n-m\right)\sdot\left(n+m\right)\right]=m^2</math> |
|style="text-align:right;"|וגם נאמר שכל מספר הנה מרובעו יוסיף על מושטח שתי פאותיו הרחוקות מרחק שוה תהיינה מה שתהיינה אחת מהם באחרת כמרובע מספר המדרגות אשר ביניהם | |style="text-align:right;"|וגם נאמר שכל מספר הנה מרובעו יוסיף על מושטח שתי פאותיו הרחוקות מרחק שוה תהיינה מה שתהיינה אחת מהם באחרת כמרובע מספר המדרגות אשר ביניהם | ||
|- | |- | ||
| Line 98: | Line 123: | ||
|- | |- | ||
| | | | ||
| − | *For every number, | + | *For every number, the interval of the terms between it and its double: |
:If one takes it as the first of the terms - it is the same as the number plus one: <math>\scriptstyle2n-\left(n-1\right)=n+1</math> | :If one takes it as the first of the terms - it is the same as the number plus one: <math>\scriptstyle2n-\left(n-1\right)=n+1</math> | ||
| − | |style="text-align:right;"|וכל מספר הנה מרחקו | + | |style="text-align:right;"|וכל מספר הנה מרחקו ב{{#annot:term|1344,1578|TrIG}}מדרגות{{#annotend:TrIG}} מכפלו אם כשתקח אותו ראשון למדרגות הנה הוא כמו מספרו ותוספת אחד |
|- | |- | ||
| | | | ||
| Line 119: | Line 144: | ||
|- | |- | ||
| | | | ||
| − | *For every number, | + | *For every number, the interval between it and its thrice is the same as the measure of its units multiplied by two: |
|style="text-align:right;"|וכל מספר מרחקו משלשה כפליו הנה הוא בכמו שיעור אחדיו מוכים בשנים | |style="text-align:right;"|וכל מספר מרחקו משלשה כפליו הנה הוא בכמו שיעור אחדיו מוכים בשנים | ||
|- | |- | ||
| | | | ||
| − | :either | + | :either with the addition of one: <math>\scriptstyle3n-\left(n-1\right)=2n+1</math> |
|style="text-align:right;"|אם בתוספת אחד | |style="text-align:right;"|אם בתוספת אחד | ||
|- | |- | ||
| | | | ||
| − | :or without the addition of | + | :or without the addition of one, as is known: <math>\scriptstyle3n-n=2n</math> |
|style="text-align:right;"|אם בזולת תוספת אחד כפי מה שידעת אותו | |style="text-align:right;"|אם בזולת תוספת אחד כפי מה שידעת אותו | ||
|- | |- | ||
| | | | ||
| − | :Such as the two, whose | + | :Such as the two, whose interval between it and 6, which is its thrice, is as its multiplication by two plus one: <math>\scriptstyle{\color{blue}{\left(3\sdot2\right)-1=\left(2\sdot2\right)+1}}</math> |
:or without the addition: <math>\scriptstyle{\color{blue}{\left(3\sdot2\right)-2=2\sdot2}}</math> | :or without the addition: <math>\scriptstyle{\color{blue}{\left(3\sdot2\right)-2=2\sdot2}}</math> | ||
|style="text-align:right;"|כמו השנים אשר מרחקם מו' שהוא ג' דמיוניו הוא כמספר הכאתו בשנים בתוספת ובזולת תוספת | |style="text-align:right;"|כמו השנים אשר מרחקם מו' שהוא ג' דמיוניו הוא כמספר הכאתו בשנים בתוספת ובזולת תוספת | ||
|- | |- | ||
| | | | ||
| − | *For every number, its | + | *For every number, its interval between it and its fourfold is the same as its multiplication by three plus [one]: <math>\scriptstyle4n-\left(n-1\right)=3n+1</math> |
|style="text-align:right;"|וכל מספר מרחקו מד' כפליו הוא כהכאתו בג' מהמספר בתוספת | |style="text-align:right;"|וכל מספר מרחקו מד' כפליו הוא כהכאתו בג' מהמספר בתוספת | ||
|- | |- | ||
| Line 154: | Line 179: | ||
|- | |- | ||
| | | | ||
| − | : | + | ::Such as: |
| + | ::*<math>\scriptstyle{\color{blue}{2^2-2=2\sdot1}}</math> | ||
|style="text-align:right;"|כמו הכאת השנים באחד אשר הוא מרחקו ממרובעו כשלא נוסיף | |style="text-align:right;"|כמו הכאת השנים באחד אשר הוא מרחקו ממרובעו כשלא נוסיף | ||
|- | |- | ||
| | | | ||
| − | : | + | ::*<math>\scriptstyle{\color{blue}{3^2-3=3\sdot2}}</math> |
|style="text-align:right;"|והכאת השלשה בשנים אשר הוא מרחק השלשה ממרובעו בשלא נוסיף | |style="text-align:right;"|והכאת השלשה בשנים אשר הוא מרחק השלשה ממרובעו בשלא נוסיף | ||
|- | |- | ||
| | | | ||
| − | * <math>\scriptstyle n^2=\left[\left(n+1\right)\sdot\left(n-1\right)\right]+1</math | + | *For every number, its square is equal to the product of [the number] plus one by the preceding number, plus one <math>\scriptstyle n^2=\left[\left(n+1\right)\sdot\left(n-1\right)\right]+1</math> |
|style="text-align:right;"|וכמו כן כל מספר הנה מרובעו שוה להכאתו בתוספת אחד במספר אשר לפניו ותוספת אחד | |style="text-align:right;"|וכמו כן כל מספר הנה מרובעו שוה להכאתו בתוספת אחד במספר אשר לפניו ותוספת אחד | ||
|- | |- | ||
| | | | ||
| − | : | + | ::Such as: |
| + | ::*<math>\scriptstyle{\color{blue}{2^2=\left(3\sdot1\right)+1}}</math> | ||
|style="text-align:right;"|כמו מרובע השנים אשר הוא שוה להכאת השלשה באחד ותוספת אחד | |style="text-align:right;"|כמו מרובע השנים אשר הוא שוה להכאת השלשה באחד ותוספת אחד | ||
|- | |- | ||
| | | | ||
| − | : | + | ::*<math>\scriptstyle{\color{blue}{3^2=\left(4\sdot2\right)+1}}</math> |
|style="text-align:right;"|ומרובע השלשה אשר הוא שוה להכאת הארבעה בשנים ותוספת אחד | |style="text-align:right;"|ומרובע השלשה אשר הוא שוה להכאת הארבעה בשנים ותוספת אחד | ||
|- | |- | ||
| | | | ||
| − | * <math>\scriptstyle\left[n\sdot\left(n-1\right)\right]-\left(n-1\right)=\left(n-1\right)^2</math> | + | *For every number, the interval between it and its product by the preceding number is as the square of the preceding number, when it is not included <math>\scriptstyle\left[n\sdot\left(n-1\right)\right]-\left(n-1\right)=\left(n-1\right)^2</math> |
|style="text-align:right;"|וכל מספר הנה מרחקו מהכאתו במספר אשר לפניו הוא כמו מרבע המספר אשר לפניו כשלא יתוסף | |style="text-align:right;"|וכל מספר הנה מרחקו מהכאתו במספר אשר לפניו הוא כמו מרבע המספר אשר לפניו כשלא יתוסף | ||
|- | |- | ||
| | | | ||
| − | : | + | ::For example: |
| + | ::*<math>\scriptstyle{\color{blue}{\left(3\sdot2\right)-2=2^2}}</math> | ||
|style="text-align:right;"|המשל בזה שמרחק השלשה מהכאתו בשלשה הוא כמו מספר מרבע השנים | |style="text-align:right;"|המשל בזה שמרחק השלשה מהכאתו בשלשה הוא כמו מספר מרבע השנים | ||
|- | |- | ||
| | | | ||
| − | : | + | ::*<math>\scriptstyle{\color{blue}{\left(4\sdot3\right)-3=3^2}}</math> |
|style="text-align:right;"|ומרחק הארבעה מהכאתו בשלשה הוא כמו מספר מרבע שלשה רצוני בזה כשלא יתוסף | |style="text-align:right;"|ומרחק הארבעה מהכאתו בשלשה הוא כמו מספר מרבע שלשה רצוני בזה כשלא יתוסף | ||
|- | |- | ||
| | | | ||
| − | * <math>\scriptstyle\left[n\sdot\left(n+1\right)\right]-n=n^2</math> | + | *For every number, the interval between it and its product by the succeeding number is as its square <math>\scriptstyle\left[n\sdot\left(n+1\right)\right]-n=n^2</math> |
|style="text-align:right;"|וכל מספר הנה מרחקו מהכאתו במספר אשר אחריו כמו מספר מרובעו | |style="text-align:right;"|וכל מספר הנה מרחקו מהכאתו במספר אשר אחריו כמו מספר מרובעו | ||
|- | |- | ||
| Line 198: | Line 226: | ||
|- | |- | ||
| | | | ||
| − | * <math>\scriptstyle n^3-n=\left[n\sdot\left(n+1\right)\right]\sdot\left(n-1\right)</math> | + | *For every number, the [number of the] terms between it and its cube is as its product by the succeeding number multiplied by the preceding number <math>\scriptstyle n^3-n=\left[n\sdot\left(n+1\right)\right]\sdot\left(n-1\right)</math> |
|style="text-align:right;"|וכל מספר הנה בינו ובין מעקבו מהמדרגות כמו הכאתו באשר ימשך אליו אחר כן הכאת זה כלו באשר לפניו | |style="text-align:right;"|וכל מספר הנה בינו ובין מעקבו מהמדרגות כמו הכאתו באשר ימשך אליו אחר כן הכאת זה כלו באשר לפניו | ||
|- | |- | ||
| | | | ||
| − | : | + | ::Such as: |
| + | ::*<math>\scriptstyle{\color{blue}{2^3-2=\left(2\sdot3\right)\sdot1}}</math> | ||
|style="text-align:right;"|כמו שנים בג' אחר כן באחד | |style="text-align:right;"|כמו שנים בג' אחר כן באחד | ||
|- | |- | ||
| | | | ||
| − | : | + | ::*<math>\scriptstyle{\color{blue}{3^3-3=\left(3\sdot4\right)\sdot2}}</math> |
|style="text-align:right;"|ושלשה בארבעה אחר כן בשנים | |style="text-align:right;"|ושלשה בארבעה אחר כן בשנים | ||
|- | |- | ||
| Line 225: | Line 254: | ||
|- | |- | ||
| | | | ||
| − | : | + | ::For example: <math>\scriptstyle{\color{blue}{2\sdot10^2+2=202=81+121=9^2+11^2}}</math> |
|style="text-align:right;"|המשל בזה פי' הכאת עשרה בעצמו בתוספת שנים והוא מאתים ושנים הנה הוא שוה להכאת תשעה בעצמו והוא שמנים ואחד ולהכאת אחד עשר בעצמו והוא מאה ועשרים ואחד | |style="text-align:right;"|המשל בזה פי' הכאת עשרה בעצמו בתוספת שנים והוא מאתים ושנים הנה הוא שוה להכאת תשעה בעצמו והוא שמנים ואחד ולהכאת אחד עשר בעצמו והוא מאה ועשרים ואחד | ||
|- | |- | ||
| | | | ||
| − | * <math>\scriptstyle2n^2+8=\left(n-2\right)^2+\left(n+2\right)^2</math> | + | *For every number, its square, when it is doubled and eight is added to it, it is equal to the sum of the two squares of its two secondary sides <math>\scriptstyle2n^2+8=\left(n-2\right)^2+\left(n+2\right)^2</math> |
|style="text-align:right;"|וכל מספר הנה מרובעו כאשר יכפל ויתוסף עליו שמנה הוא שוה למקובץ שני מרובעי שתי פאותיו השניות | |style="text-align:right;"|וכל מספר הנה מרובעו כאשר יכפל ויתוסף עליו שמנה הוא שוה למקובץ שני מרובעי שתי פאותיו השניות | ||
|- | |- | ||
| | | | ||
| − | : | + | ::For example: <math>\scriptstyle{\color{blue}{2\sdot10^2+8=208=8^2+12^2}}</math> |
|style="text-align:right;"|המשל בזה עשרה אשר מרובעו כשיעשה בו זה יהיה מאתים ושמנה והוא שוה להכאת שמנה בעצמו | |style="text-align:right;"|המשל בזה עשרה אשר מרובעו כשיעשה בו זה יהיה מאתים ושמנה והוא שוה להכאת שמנה בעצמו | ||
|- | |- | ||
| | | | ||
| − | * <math>\scriptstyle2n^2+18=\left(n-3\right)^2+\left(n+3\right)^2</math> | + | *For every number, whose square is doubled and eighteen is added to it, it is equal to [the sum of] the two squares of its tertiary sides <math>\scriptstyle2n^2+18=\left(n-3\right)^2+\left(n+3\right)^2</math> |
|style="text-align:right;"|וכל מספר אשר יכפל מרובעו ויתוסף עליו שמנה עשר הנה יהיה שוה לשני מרבעי פאותיו השלישיות | |style="text-align:right;"|וכל מספר אשר יכפל מרובעו ויתוסף עליו שמנה עשר הנה יהיה שוה לשני מרבעי פאותיו השלישיות | ||
|- | |- | ||
| | | | ||
| − | : | + | ::For example: <math>\scriptstyle{\color{blue}{2\sdot10^2+18=218=7^2+13^2}}</math> |
|style="text-align:right;"|המשל בזה מאתים ושמנה עשר אשר הוא שוה להכאת שבעה בעצמו ושלשה עשר בעצמו | |style="text-align:right;"|המשל בזה מאתים ושמנה עשר אשר הוא שוה להכאת שבעה בעצמו ושלשה עשר בעצמו | ||
|- | |- | ||
| | | | ||
| − | * <math>\scriptstyle2n^2+32=\left(n-4\right)^2+\left(n+4\right)^2</math> | + | *For the two quarterly sides the addition is thirty two <math>\scriptstyle2n^2+32=\left(n-4\right)^2+\left(n+4\right)^2</math> |
|style="text-align:right;"|ואמנם בשתי פאות הרביעיות הנה התוספת שנים ושלשי‫' | |style="text-align:right;"|ואמנם בשתי פאות הרביעיות הנה התוספת שנים ושלשי‫' | ||
|- | |- | ||
| | | | ||
| − | * <math>\scriptstyle2n^2+50=\left(n-5\right)^2+\left(n+5\right)^2</math> | + | *For the two fifth sides the addition is fifty <math>\scriptstyle2n^2+50=\left(n-5\right)^2+\left(n+5\right)^2</math> |
|style="text-align:right;"|ובשתי פאות החמישיות חמשים | |style="text-align:right;"|ובשתי פאות החמישיות חמשים | ||
|- | |- | ||
| Line 269: | Line 298: | ||
|- | |- | ||
| | | | ||
| − | : | + | ::For example: <math>\scriptstyle{\color{blue}{2\sdot10^2+4=204=\left(9\sdot8\right)+\left(11\sdot12\right)}}</math> |
|style="text-align:right;"|המשל בזה מאתים וארבעה אשר הוא שוה להכאת תשעה בשמנה ואחד עשר בשנים עשר | |style="text-align:right;"|המשל בזה מאתים וארבעה אשר הוא שוה להכאת תשעה בשמנה ואחד עשר בשנים עשר | ||
|- | |- | ||
| Line 301: | Line 330: | ||
|- | |- | ||
| | | | ||
| − | : | + | ::For example: <math>\scriptstyle{\color{blue}{2\sdot10^2+6=206=\left(9\sdot7\right)+\left(11\sdot13\right)}}</math> |
|style="text-align:right;"|המשל בזה מאתים וששה אשר הוא שוה להכאת תשעה בשבעה ואחד עשר בשלשה עשר | |style="text-align:right;"|המשל בזה מאתים וששה אשר הוא שוה להכאת תשעה בשבעה ואחד עשר בשלשה עשר | ||
|- | |- | ||
| Line 313: | Line 342: | ||
|- | |- | ||
| | | | ||
| − | : | + | ::For example: <math>\scriptstyle{\color{blue}{2\sdot10^2+16=216=\left(8\sdot6\right)+\left(12\sdot14\right)}}</math> |
|style="text-align:right;"|המשל בזה מקובץ מושטחי שמנה בששה ושנים עשר בארבעה עשריו הנה זה מאתים וששה עשר | |style="text-align:right;"|המשל בזה מקובץ מושטחי שמנה בששה ושנים עשר בארבעה עשריו הנה זה מאתים וששה עשר | ||
|- | |- | ||
| Line 343: | Line 372: | ||
=== Arithmetic Progression === | === Arithmetic Progression === | ||
| − | + | | | |
| + | |- | ||
| + | | | ||
|style="text-align:right;"|<big>ונתחיל</big> אליך בסגלות המספרים הנמשכים המשכם הטבעי | |style="text-align:right;"|<big>ונתחיל</big> אליך בסגלות המספרים הנמשכים המשכם הטבעי | ||
|- | |- | ||
| Line 402: | Line 433: | ||
|- | |- | ||
| | | | ||
| − | ==== | + | ==== Sums ==== |
| | | | ||
| Line 431: | Line 462: | ||
:<math>\scriptstyle\sum_{k=m}^n k=\left(n+m\right)\sdot\left[\frac{1}{2}\sdot\left(n-m+1\right)\right]</math> | :<math>\scriptstyle\sum_{k=m}^n k=\left(n+m\right)\sdot\left[\frac{1}{2}\sdot\left(n-m+1\right)\right]</math> | ||
:<math>\scriptstyle\sum_{k=m}^n k=\left[\frac{1}{2}\sdot\left(n+m\right)\right]\sdot\left(n-m+1\right)</math> | :<math>\scriptstyle\sum_{k=m}^n k=\left[\frac{1}{2}\sdot\left(n+m\right)\right]\sdot\left(n-m+1\right)</math> | ||
| − | |style="text-align:right;"|וגם כן הנה מקובץ כל שתי קצוות מבעלי הדרגה וסדר הן שתהיינה מן האחד או מן זולתו כאשר הוכה בחצי המדרגות או הוכה חציו בכל המדרגות הנה יהיה מה שיתקבץ כמו כלל מקובץ אותם המדרגות | + | |style="text-align:right;"|וגם כן הנה מקובץ כל שתי {{#annot:term|1902,2120|xhee}}קצוות{{#annotend:xhee}} מבעלי הדרגה וסדר הן שתהיינה מן האחד או מן זולתו כאשר הוכה בחצי המדרגות או הוכה חציו בכל המדרגות הנה יהיה מה שיתקבץ כמו כלל מקובץ אותם המדרגות |
|- | |- | ||
| | | | ||
| Line 514: | Line 545: | ||
:Also, all the successive numbers, when they are summed together, the first sum is as the consecutive term, the second sum is one and a half times the consecutive term, the third sum is twice the consecutive term and so on endlessly. | :Also, all the successive numbers, when they are summed together, the first sum is as the consecutive term, the second sum is one and a half times the consecutive term, the third sum is twice the consecutive term and so on endlessly. | ||
:<math>\scriptstyle\sum_{i=1}^n i=\frac{n}{2}\sdot\left(n+1\right)</math> | :<math>\scriptstyle\sum_{i=1}^n i=\frac{n}{2}\sdot\left(n+1\right)</math> | ||
| − | |style="text-align:right;"|<big>וגם כן</big> הנה כל המספרים הנמשכים יקובצו זה הקבוץ הנה המקובץ הראשון יהיה כמו המספר הנמשך אליו והמקובץ השני דמיון וחצי מהמספר הנמשך אליו והמקובץ השלישי כפל המספר הנמשך אליו וכמו כן אל בלתי תכלית | + | |style="text-align:right;"|<big>וגם כן</big> הנה כל המספרים הנמשכים יקובצו זה הקבוץ הנה המקובץ הראשון יהיה כמו המספר הנמשך אליו והמקובץ השני {{#annot:sesquialter|125|JGKv}}דמיון וחצי{{#annotend:JGKv}} מהמספר הנמשך אליו והמקובץ השלישי כפל המספר הנמשך אליו וכמו כן אל בלתי תכלית |
|- | |- | ||
| | | | ||
| Line 536: | Line 567: | ||
*Among the properties relating to the sum: when summing the successive odds, beginning from one, then summing the successive evens, [beginning] from two, by their numbers, the first sum of the evens is one and a half times the first sum of the odds, the second sum is one and a third times, the third sum is one and a quarter times - each sum exceeds by a part that is denominated by the number of terms. | *Among the properties relating to the sum: when summing the successive odds, beginning from one, then summing the successive evens, [beginning] from two, by their numbers, the first sum of the evens is one and a half times the first sum of the odds, the second sum is one and a third times, the third sum is one and a quarter times - each sum exceeds by a part that is denominated by the number of terms. | ||
:<math>\scriptstyle\sum_{i=1}^n 2i=\left(1+\frac{1}{n}\right)\sdot\left[\sum_{i=1}^n \left(2i-1\right)\right]</math> | :<math>\scriptstyle\sum_{i=1}^n 2i=\left(1+\frac{1}{n}\right)\sdot\left[\sum_{i=1}^n \left(2i-1\right)\right]</math> | ||
| − | |style="text-align:right;"|<big>ומהסגלות</big> הנתלות בקבוץ שאתה כאשר תקבץ נפרדים נמשכים מתחילים מן האחד ותקבץ אחריהם זוגות נמשכים מן השנים כמספרם הנה המקובץ הראשון מהזוגות יהיה דמיון וחצי המקובץ הראשון מהנפרדים והמקובץ השני דמיון ושלישיתו והמקובץ השלישי דמיון ורביעיתו ויהיה כל מקובץ יוסיף בחלק נקרא על שם מספר מדרגתו ויהיה מספרו מספר מדרגתו | + | |style="text-align:right;"|<big>ומהסגלות</big> הנתלות בקבוץ שאתה כאשר תקבץ נפרדים נמשכים מתחילים מן האחד ותקבץ אחריהם {{#annot:term|63,1333|6NUn}}זוגות{{#annotend:6NUn}} נמשכים מן השנים כמספרם הנה המקובץ הראשון מהזוגות יהיה דמיון וחצי המקובץ הראשון מהנפרדים והמקובץ השני {{#annot:sesquitertian|125|6trp}}דמיון ושלישיתו{{#annotend:6trp}} והמקובץ השלישי {{#annot:sesquiquartan|125|AkWv}}דמיון ורביעיתו{{#annotend:AkWv}} ויהיה כל מקובץ יוסיף בחלק נקרא על שם מספר מדרגתו ויהיה מספרו מספר מדרגתו |
|- | |- | ||
| | | | ||
| Line 545: | Line 576: | ||
| | | | ||
::*<math>\scriptstyle{\color{blue}{2+4+6=\left(1+\frac{1}{3}\right)\sdot\left(1+3+5\right)}}</math> | ::*<math>\scriptstyle{\color{blue}{2+4+6=\left(1+\frac{1}{3}\right)\sdot\left(1+3+5\right)}}</math> | ||
| − | |style="text-align:right;"|וכאשר תוסיף בזה ששה ובזה חמשה יהיה זה דמיון ושליש זה | + | |style="text-align:right;"|וכאשר תוסיף בזה ששה ובזה חמשה יהיה זה {{#annot:sesquitertian|125|o1rS}}דמיון ושליש{{#annotend:o1rS}} זה |
|- | |- | ||
| Line 557: | Line 588: | ||
=== Types of Numbers === | === Types of Numbers === | ||
| − | |style="text-align:right;"|ונשוב | + | | |
| + | |- | ||
| + | |Returning to the presentation of the properties of the first categorization of the number from the aspect of the quality of its divisibility to equals and unequals, which are the even and the odd. | ||
| + | |style="text-align:right;"|<big>ונשוב</big> עתה אל הבאת סגלות החלוקה הראשונה מהמספר מצד איכות החלקו אל שוים ובלתי שוים והוא הזוג והנפרד | ||
|- | |- | ||
| − | | | + | |What was discussed concerning it in the book of ''the Elements'' will also be presented. |
| − | |style="text-align:right;"|ועוד נביא מה שדובר בו | + | |style="text-align:right;"|ועוד נביא מה שדובר בו בספר היסודות |
|- | |- | ||
| − | | | + | |The natural successive odds and evens are subject to association acquired from their type: |
| − | |style="text-align:right;"|וכבר יפול ביניהם | + | |style="text-align:right;"|וכבר יפול ביניהם שתוף נקנה מסוגם במה שימשך מהנפרדים והזוגו' {{#annot:term|2574,2576|F8FS}}המשכות טבעי{{#annotend:F8FS}} המשכות מיני המספר |
|- | |- | ||
| − | | | + | |The terms exceed by one excess |
| − | |style="text-align:right;"|וזה כלו שתהיינה | + | |style="text-align:right;"|וזה כלו שתהיינה ה{{#annot:term|1344,1578|NEbn}}מדרגות{{#annotend:NEbn}} עודפות בהעדף אחד |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|אם העדף ההמשכות הטבעי למיני המספר הנה באחד אחד | + | *Either by the excess of the natural succession of the types of number, which is one by one. |
| + | |style="text-align:right;"|אם העדף {{#annot:term|2574,2576|Kg5J}}ההמשכות הטבעי{{#annotend:Kg5J}} למיני המספר הנה באחד אחד | ||
|- | |- | ||
| | | | ||
| + | *Or by the excess of the natural succession of the odds and the evens, which is two by two. | ||
|style="text-align:right;"|ואם העדף הנפרדים והזוגות הנמשכים בטבע הנה בשנים שנים | |style="text-align:right;"|ואם העדף הנפרדים והזוגות הנמשכים בטבע הנה בשנים שנים | ||
|- | |- | ||
| | | | ||
| − | *odd | + | :*for every odd number, when one is added to it, it becomes even. |
| + | ::[<math>\scriptstyle\left(2n-1\right)+1=2n</math>] | ||
|style="text-align:right;"|לפי שכל נפרד כאשר יתוסף עליו אחד יהיה זוג | |style="text-align:right;"|לפי שכל נפרד כאשר יתוסף עליו אחד יהיה זוג | ||
|- | |- | ||
| | | | ||
| − | * | + | :*When one is further added to it, it becomes odd. |
| + | ::[<math>\scriptstyle\left[\left(2n-1\right)+1\right]+1=2n+1=\left[2\sdot\left(n+1\right)\right]-1</math>] | ||
|style="text-align:right;"|אחר כן כשיתוסף עליו אחד אחד יהיה נפרד | |style="text-align:right;"|אחר כן כשיתוסף עליו אחד אחד יהיה נפרד | ||
|- | |- | ||
| | | | ||
| − | * | + | :*Then, when one is added to it, it becomes even. |
| + | ::<math>\scriptstyle\left[\left[\left(2n-1\right)+1\right]+1\right]+1=2\sdot\left(n+1\right)</math> | ||
|style="text-align:right;"|אח"כ כשיתוסף עליו אחד יהיה זוג | |style="text-align:right;"|אח"כ כשיתוסף עליו אחד יהיה זוג | ||
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle\left[\left[2\sdot\left(n+1\right)\right]-1\right]-\left(2n-1\right)=2</math> | + | :*Between the odd and the following odd there are two. |
| + | ::<math>\scriptstyle\left[\left[2\sdot\left(n+1\right)\right]-1\right]-\left(2n-1\right)=2</math> | ||
|style="text-align:right;"|ויהיה בין הנפרד והנפרד אשר ילוה אליו שנים | |style="text-align:right;"|ויהיה בין הנפרד והנפרד אשר ילוה אליו שנים | ||
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle\left[2\sdot\left(n+1\right)\right]-2n=2</math> | + | :*Between the even and the following even there are two. |
| + | ::<math>\scriptstyle\left[2\sdot\left(n+1\right)\right]-2n=2</math> | ||
|style="text-align:right;"|ובין הזוג והזוג אשר ילוה אליו שנים | |style="text-align:right;"|ובין הזוג והזוג אשר ילוה אליו שנים | ||
|- | |- | ||
| − | | | + | | |
| − | + | *It follows necessarily that every mean of the odd terms in the natural succession and the even terms in this succession is half the sum of the two sides, whichever they are. | |
| + | :<math>\scriptstyle n=\frac{1}{2}\sdot\left[\left(n-m\right)+\left(n+m\right)\right]</math> | ||
|style="text-align:right;"|ויחויב שיהיה כל אמצעי במדרגות הנפרדים אשר על ההמשך הטבעי ומדרגות הזוגות אשר על זה ההמשך כמו חצי מקובץ איזה שתי פאות שתהיינה | |style="text-align:right;"|ויחויב שיהיה כל אמצעי במדרגות הנפרדים אשר על ההמשך הטבעי ומדרגות הזוגות אשר על זה ההמשך כמו חצי מקובץ איזה שתי פאות שתהיינה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|לפי שהן פאות זה האמצעי בעצמו בסדור | + | :Since they are the sides of this mean itself in the natural succession of the numbers. |
| + | |style="text-align:right;"|לפי שהן פאות זה האמצעי בעצמו {{#annot:term|1402,2576|TmGX}}בסדור הטבעי למספר{{#annotend:TmGX}} | ||
|- | |- | ||
| − | | | + | | |
| − | + | *The sum of every two means is as the sum of every two sides. | |
| + | :<math>\scriptstyle n+\left(n+1\right)=\left(n-m\right)+\left[\left(n+1\right)+m\right]</math> | ||
|style="text-align:right;"|וכל שני אמצעיים מקובצים כמו כל שתי פאות מקובצות | |style="text-align:right;"|וכל שני אמצעיים מקובצים כמו כל שתי פאות מקובצות | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|לפי שבאלה השני אמצעיים תהיינה שתי פאות למספר הנופל בסדר | + | :Since these two means have two sides of the number that falls between them in the succession of the natural numbers, it follows necessarily that their sum is equal to the sum of these two other sides, as explained above. |
| + | |style="text-align:right;"|לפי שבאלה השני אמצעיים תהיינה שתי פאות למספר הנופל בסדר המספרים הטבעיים ביניהם והנה יחויב שיהיה שוה מקובצם למקובץ אלה שתי הפאות האחרות כפי מה שקדם באורו | ||
|- | |- | ||
| | | | ||
| + | :This property does not applied between the successive odds and the successive evens alone, but between all the numbers that are exceeding by equality. | ||
|style="text-align:right;"|ואין זה הענין נוהג בין הנפרדים הנמשכים והזוגות הנמשכי' לבד אבל בין כל המספרים העודפים על השווי | |style="text-align:right;"|ואין זה הענין נוהג בין הנפרדים הנמשכים והזוגות הנמשכי' לבד אבל בין כל המספרים העודפים על השווי | ||
|- | |- | ||
| | | | ||
| + | :Hence, this property is found also in the succession of the terms of the types of the odd numbers | ||
|style="text-align:right;"|ולזה תמצא זאת הסגלה ג"כ בסדר מדרגות מני הנפרד | |style="text-align:right;"|ולזה תמצא זאת הסגלה ג"כ בסדר מדרגות מני הנפרד | ||
|- | |- | ||
| − | | | + | |Thus, this association should be stated before all the properties. |
|style="text-align:right;"|והנה זה ההשתתפות ראוי שנאמר אותו קודם הסגלות | |style="text-align:right;"|והנה זה ההשתתפות ראוי שנאמר אותו קודם הסגלות | ||
|- | |- | ||
| − | | | + | |Now, we will concentrate in mentioning the properties |
|style="text-align:right;"|ונתבודד עתה בזכרון הסגלות | |style="text-align:right;"|ונתבודד עתה בזכרון הסגלות | ||
|- | |- | ||
| Line 623: | Line 670: | ||
==== Odd Number ==== | ==== Odd Number ==== | ||
| − | | | + | | |
|- | |- | ||
| − | | | + | |Starting with the properties of the odd number |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<big>ונתחיל</big> בסגלות ה{{#annot:term|65,1336|pq13}}נפרד{{#annotend:pq13}} |
|- | |- | ||
| − | | | + | |The known and mentioned properties are that it does not consist of evens at all and not from an even number of odd numbers. |
| + | :[odd ≠ even × even] | ||
| + | :[odd ≠ even × odd] | ||
| + | |style="text-align:right;"|ונאמר אמנם הסגלות הידועות והנזכרות מאשר הוא לא יתרכב מזוגות כלל ולא מ{{#annot:term|65,1336|R19n}}נפרדים{{#annotend:R19n}} במספר זוג | ||
| + | |- | ||
| + | |There is no number of its type in it, whose remainder is of its type | ||
| + | :[odd − odd ≠ odd] | ||
|style="text-align:right;"|ושלא ימצא בו מסוגו מספר ישאיר מה שאחריו מסוגו | |style="text-align:right;"|ושלא ימצא בו מסוגו מספר ישאיר מה שאחריו מסוגו | ||
|- | |- | ||
| − | | | + | |There is no number of its parallel type in it, whose remainder is of its parallel type |
| + | :[odd − even ≠ even] | ||
|style="text-align:right;"|ושלא ימצא בו מסוג מקבילו מספר ישאיר מה שאחריו מסוג מקבילו | |style="text-align:right;"|ושלא ימצא בו מסוג מקבילו מספר ישאיר מה שאחריו מסוג מקבילו | ||
| − | |||
|- | |- | ||
| − | | | + | |What is said about whatever is applied to these properties in the book of the ''Elements'' is sufficient. |
| − | |style="text-align:right;"|ונדבר מסגלותיו סגלות נתלות בסדור היותם | + | |style="text-align:right;"|ומה שירוץ מרוצת אלו הסגלות הנה נסתפק במה שנאמר בספר היסודות |
| + | |- | ||
| + | |Its properties that will be discussed are the properties related to the sequence, their being successive by the way of succession | ||
| + | |style="text-align:right;"|ונדבר מסגלותיו סגלות נתלות בסדור היותם נמשכים על דרך ההמשך | ||
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle\sum_{i=1}^n \left(2i-1\right)=m^2</math> | + | *Among its properties: its sum by the way of succession, [starting] from the one, is always a square. |
| − | |style="text-align:right;"|ומסגלותיו שמקובציו על דרך ההמשך מן האחד יהיה מרובע לעולם | + | :<math>\scriptstyle\sum_{i=1}^n \left(2i-1\right)=m^2</math> |
| + | |style="text-align:right;"|<big>ומסגלותיו</big> שמקובציו על דרך ההמשך מן האחד יהיה מרובע לעולם | ||
|- | |- | ||
| | | | ||
| − | : | + | ::Such as: |
| + | ::*<math>\scriptstyle{\color{blue}{1+3}}</math> | ||
|style="text-align:right;"|כמו האחד והשלשה | |style="text-align:right;"|כמו האחד והשלשה | ||
|- | |- | ||
| | | | ||
| − | : | + | ::*<math>\scriptstyle{\color{blue}{1+3+5}}</math> |
|style="text-align:right;"|ואח"כ האחד והשלשה והחמשה | |style="text-align:right;"|ואח"כ האחד והשלשה והחמשה | ||
|- | |- | ||
| | | | ||
| − | : | + | ::*<math>\scriptstyle{\color{blue}{1+3+5+7}}</math> |
|style="text-align:right;"|ואח"כ האחד והשלשה והחמשה והשבעה | |style="text-align:right;"|ואח"כ האחד והשלשה והחמשה והשבעה | ||
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle\sum_{i=1}^n \left(2i-1\right)=n^2</math> | + | *Among its properties: the side of each of these squares is the number of the terms |
| − | |style="text-align:right;"|ומסגלותיו שכל מרבע מאלו הנה צלעו מספר המדרגות | + | :<math>\scriptstyle\sum_{i=1}^n \left(2i-1\right)=n^2</math> |
| + | |style="text-align:right;"|<big>ומסגלותיו</big> שכל מרבע מאלו הנה צלעו מספר המדרגות | ||
|- | |- | ||
| | | | ||
| − | : | + | ::Such as: |
| + | ::*the four, which is the sum of two terms and its root is two | ||
| + | :::<math>\scriptstyle{\color{blue}{1+3=\sum_{i=1}^2 \left(2i-1\right)=4=2^2}}</math> | ||
|style="text-align:right;"|כמו הארבעה והוא מקובץ שתי המדרגות והנה גדרו שנים | |style="text-align:right;"|כמו הארבעה והוא מקובץ שתי המדרגות והנה גדרו שנים | ||
|- | |- | ||
| | | | ||
| − | : | + | ::*the nine, which is the sum of three terms and its root is three |
| + | :::<math>\scriptstyle{\color{blue}{1+3+5=\sum_{i=1}^3 \left(2i-1\right)=9=3^2}}</math> | ||
|style="text-align:right;"|והתשעה והוא מקובץ שלשה מדרגות והנה גדרו שלשה | |style="text-align:right;"|והתשעה והוא מקובץ שלשה מדרגות והנה גדרו שלשה | ||
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle a_n=2n-1</math> | + | *Among its properties: when wishing to know the value of a number whose position is known, starting from the one - multiplying the number of the term by two, then subtracting one. |
| − | : | + | :<math>\scriptstyle a_n=2n-1</math> |
| − | |style="text-align:right;"|ומסגלותיו שאתה כשתרצה לדעת הגעת מספר יפול | + | ::For example: <math>\scriptstyle{\color{blue}{a_{10}=\left(2\sdot10\right)-1=20-1=19}}</math> |
| + | |style="text-align:right;"|<big>ומסגלותיו</big> שאתה כשתרצה לדעת הגעת מספר יפול ב{{#annot:term|1344,1578|6pVQ}}מדרגה{{#annotend:6pVQ}} ידועה מן האחד כמו העשירית והאחת עשרה דרך משל וזולת זה הנה תכה מספר המדרגה ותהיה העשירית ומספרה שני עשרה בשנים ויהיו עשרים והנה תגרע מהם אחד ויהיו תשעה עשר והנה הוא מספר המדרגה העשירית | ||
|- | |- | ||
| | | | ||
| + | :The property of the mean and the two means, with the two sides, is as known. | ||
|style="text-align:right;"|ואמנם ענין האמצעי והשני אמצעיים עם השתי פאות הנה הוא ממה שידעת אותו | |style="text-align:right;"|ואמנם ענין האמצעי והשני אמצעיים עם השתי פאות הנה הוא ממה שידעת אותו | ||
|- | |- | ||
| | | | ||
| − | * | + | *Among its properties: each of the units returns every sixth term. |
| − | |style="text-align:right;"|ומסגלותיו שכל אחד מן האחדים ישוב בששי ממנו אליו | + | |style="text-align:right;"|<big>ומסגלותיו</big> שכל אחד מן האחדים ישוב בששי ממנו אליו |
|- | |- | ||
| | | | ||
| − | : | + | ::For example: |
| + | ::<math>\scriptstyle a_{1+5n}=10m+1</math> | ||
| + | ::*<math>\scriptstyle{\color{blue}{a_{1+5}=a_6=1}}{\color{red}{\mathbf{1}}}</math> | ||
|style="text-align:right;"|המשל בזה שהאחד ישוב בששי והוא האחד עשר | |style="text-align:right;"|המשל בזה שהאחד ישוב בששי והוא האחד עשר | ||
|- | |- | ||
| | | | ||
| − | : | + | ::*<math>\scriptstyle{\color{blue}{a_{1+5+5}=a_{11}=2}}{\color{red}{\mathbf{1}}}</math> |
|style="text-align:right;"|ועוד בששי אחר הששי והוא האחד ועשרים | |style="text-align:right;"|ועוד בששי אחר הששי והוא האחד ועשרים | ||
|- | |- | ||
| | | | ||
| − | + | ::<math>\scriptstyle a_{2+5n}=10m+3</math> | |
| − | : | + | ::*<math>\scriptstyle{\color{blue}{a_2=}}{\color{red}{\mathbf{3}}}</math> |
| − | : | + | ::*<math>\scriptstyle{\color{blue}{a_{2+5}=a_7=1}}{\color{red}{\mathbf{3}}}</math> |
|style="text-align:right;"|והשלשה ישוב לששי והוא השלשה עשר וכן על הדרך הזה | |style="text-align:right;"|והשלשה ישוב לששי והוא השלשה עשר וכן על הדרך הזה | ||
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle a_n=p\ | + | *Among its properties: for every prime odd <math>\scriptstyle a_n=p</math>, when extracting from it the interval, whose value is that number, its end <math>\scriptstyle a_{n+p}=</math> is a composite number that consists of deficient primes. |
| − | |style="text-align:right;"|ומסגלותיו שכל נפרד ראשון כאשר תוציא קוו על מספרו יכלה אל מורכב ימשכו אליו ראשונים הם חסרי‫' | + | |style="text-align:right;"|<big>ומסגלותיו</big> שכל נפרד ראשון כאשר תוציא קוו על מספרו יכלה אל מורכב ימשכו אליו ראשונים הם חסרי‫' |
|- | |- | ||
| | | | ||
| − | : | + | ::Such as: |
| + | ::*three, for the third from it is nine <math>\scriptstyle{\color{blue}{a_i=3\longrightarrow a_{i+3}=9}}</math>, which is composite. | ||
|style="text-align:right;"|כמו השלשה כי הנה השלישי ממנו והוא תשעה מורכב | |style="text-align:right;"|כמו השלשה כי הנה השלישי ממנו והוא תשעה מורכב | ||
|- | |- | ||
| | | | ||
| − | : | + | ::*five, for the fifth from it, which is 15 <math>\scriptstyle{\color{blue}{a_i=5\longrightarrow a_{i+5}=15}}</math>, is composite. |
|style="text-align:right;"|והחמשה כי הנה החמישי ממנו והוא חמשה עשר מורכב | |style="text-align:right;"|והחמשה כי הנה החמישי ממנו והוא חמשה עשר מורכב | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|וסגלה אחרת שהראשון שבמספרים הבלתי מורכבים והוא שלשה יביא כשתוציא קוו הראשון אל נגדר אחר כן לא יביא‫<ref>marg. א"ב אם רצה בזה שלא יביא אל נגדר בהקוות השני או שלא יביא אל נגדר באי זה הקוות שיהיה הוא נכון ואולם אם רצה בזה שלא יביא אל נגדר לעולם אין זה צודק כי השלשה אחר י"ג הקוויות יביא אל נגדר אצל פ"א וגם זה יצדק בחמשה ושאר המספרים כאשר תוציא קום</ref> | + | *Another property: the first of the incomposite numbers, which is three, when extracting from it the first interval of its value, it yields a number that has a root <math>\scriptstyle{\color{blue}{a_i=3\longrightarrow a_{i+3}=n^2}}</math>, but afterwards not <math>\scriptstyle{\color{blue}{a_{i+3+3}\ne m^2}}</math> |
| + | |style="text-align:right;"|<big>וסגלה</big> אחרת שהראשון שבמספרים הבלתי מורכבים והוא שלשה יביא כשתוציא קוו הראשון אל נגדר אחר כן לא יביא‫<ref>marg. א"ב אם רצה בזה שלא יביא אל נגדר בהקוות השני או שלא יביא אל נגדר באי זה הקוות שיהיה הוא נכון ואולם אם רצה בזה שלא יביא אל נגדר לעולם אין זה צודק כי השלשה אחר י"ג הקוויות יביא אל נגדר אצל פ"א וגם זה יצדק בחמשה ושאר המספרים כאשר תוציא קום</ref> | ||
| + | |- | ||
| + | | | ||
| + | :And the second [incomposite number], which is five, yields, by the second interval of that value, a number that has a root <math>\scriptstyle{\color{blue}{a_i=5\longrightarrow a_{i+5+5}=25}}</math>, but afterwards not <math>\scriptstyle{\color{blue}{a_{i+5+5+5}\ne n^2}}</math> | ||
| + | |style="text-align:right;"|והשני והוא החמשה יביא בהקוות השני אל נגדר אצל עשרים וחמשה אח"כ לא יבוא | ||
|- | |- | ||
| | | | ||
| − | : | + | :And so on. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ועל הדרך הזה |
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle a_{1+ | + | *Another property: the fourth after the first that has a root, which is one <math>\scriptstyle a_1=1=m^2</math>, has a root, which is nine <math>\scriptstyle a_{1+4}=m_1^2</math>, then the eighth after the first that has a root <math>\scriptstyle a_{1+4+8}=m_2^2</math>, then the twelfth after the second that has a root <math>\scriptstyle a_{1+4+8+12}=m_3^2</math>, then the sixteenth after the third that has a root <math>\scriptstyle a_{1+4+8+12+16}=m_4^2</math>, and so on by adding four <math>\scriptstyle1+4\sdot\sum_{i=1}^n 2i=\left(1+2n\right)^2</math> |
| − | |style="text-align:right;"|וסגלה אחרת שהרביעי אחר הנגדר הראשון והוא אחד נגדר והוא תשעה והשמיני אחר הנגדר הראשון והשנים עשר | + | |style="text-align:right;"|<big>וסגלה</big> אחרת שהרביעי אחר הנגדר הראשון והוא אחד נגדר והוא תשעה והשמיני אחר הנגדר הראשון והשנים עשר אחר הנגדר השני והששה עשר אחר הנגדר השלישי וכן בתוספת ארבעה ארבעה‫<ref>marg.א"ב למעלה קרא נגדר ראשון האחד ובכאן קרא נגדר ראשון התשעה והוא הנכון כי האחד אינו מספר ודבר למעלה דרך העברה והסדר הוא שהראשון שבנגדרים הנפרדים תשעה והשני כ"ה והשלישי מ"ט ועל הדרך הזה</ref> |
|- | |- | ||
| | | | ||
| − | + | :For every term and rank, whose value has a root, the product of the root [by itself] is equal to double the number of the rank plus one. | |
| + | :<math>\scriptstyle a_{1+4n}=m^2=\left(2\sdot4n\right)+1</math> | ||
|style="text-align:right;"|וכל בית ומדרגה שתפול בו נגדר הנה תהיה הכאת זה הנגדר שוה לכפל מספר המדרגה מוסף עליו אחד | |style="text-align:right;"|וכל בית ומדרגה שתפול בו נגדר הנה תהיה הכאת זה הנגדר שוה לכפל מספר המדרגה מוסף עליו אחד | ||
|- | |- | ||
| | | | ||
| − | : | + | ::Because, the first square number is nine, which is in the fourth term of the odd numbers: <math>\scriptstyle{\color{blue}{a_{1+4}=9=\left(2\sdot4\right)+1}}</math> |
|style="text-align:right;"|כי המספר המרובע הראשון הוא תשעה והוא במדרגה הרביעי' מן המספרים הנפרדים וכפל הארבעה שמנה והנה תוסיף עליו אחד | |style="text-align:right;"|כי המספר המרובע הראשון הוא תשעה והוא במדרגה הרביעי' מן המספרים הנפרדים וכפל הארבעה שמנה והנה תוסיף עליו אחד | ||
|- | |- | ||
| | | | ||
| − | : | + | ::And 25 is in the twelfth term from 3: <math>\scriptstyle{\color{blue}{a_{1+12}=25=\left(2\sdot12\right)+1}}</math> |
|style="text-align:right;"|והבית השנים עשר מהשלשה יפול בו חמשה ועשרים והוא שוה לכפל שנים עשר כשתוסיף עליו אחד | |style="text-align:right;"|והבית השנים עשר מהשלשה יפול בו חמשה ועשרים והוא שוה לכפל שנים עשר כשתוסיף עליו אחד | ||
|- | |- | ||
| − | | | + | |When the natural successive odds are set in a square table, properties are seen from the aspect of the shape. |
| − | |style="text-align:right;"|וכאשר נניח מהנפרדים הנמשכים בטבע לוח מרובע יראו בו סגלות מצד התמונה וכן כאשר נניח לוח משלש | + | |style="text-align:right;"|וכאשר נניח מהנפרדים הנמשכים בטבע לוח מרובע יראו בו סגלות מצד התמונה |
| + | |- | ||
| + | |Also, when a triangle table is set. | ||
| + | |style="text-align:right;"|וכן כאשר נניח לוח משלש | ||
|- | |- | ||
| | | | ||
| + | ::*Starting with a square [table] of five by five: | ||
|style="text-align:right;"|ונתחיל במרובע ונשים אותו חמשה על חמשה | |style="text-align:right;"|ונתחיל במרובע ונשים אותו חמשה על חמשה | ||
|- | |- | ||
| | | | ||
| − | {|class="wikitable" style="color: blue; text-align:center;" | + | :::{|class="wikitable" style="color: blue; text-align:center;" |
|- | |- | ||
|9||7||5||3||1 | |9||7||5||3||1 | ||
| Line 763: | Line 840: | ||
|- | |- | ||
| | | | ||
| − | *the sums of | + | *For every two symmetric diagonals, whether they are the principal diagonals of the diagram or not, the sums of both diagonals are equal. |
|style="text-align:right;"|ונאמר שכל שתי וערב ממנו יהיה קטר התמונה או לא יהיה הנה מקובץ שני קטריו הם שוים | |style="text-align:right;"|ונאמר שכל שתי וערב ממנו יהיה קטר התמונה או לא יהיה הנה מקובץ שני קטריו הם שוים | ||
|- | |- | ||
| | | | ||
| − | : | + | :::For the principal diagonals: the sum of each diagonal in this diagram is 125. |
| + | :::[<math>\scriptstyle{\color{blue}{1+13+25+37+49=125=9+17+25+33+41}}</math>] | ||
|style="text-align:right;"|אם אשר על הקטר הנה מקובץ כל אחד מהשני קטרים אשר בזאת התמונה קכ"ה | |style="text-align:right;"|אם אשר על הקטר הנה מקובץ כל אחד מהשני קטרים אשר בזאת התמונה קכ"ה | ||
|- | |- | ||
| | | | ||
| − | : | + | :::If they are not the principal diagonals, as these two symmetric diagonals: <math>\scriptstyle{\color{blue}{3+15+27=45=7+15+23}}</math> |
|style="text-align:right;"|ואם אשר אינם על הקטר הנה כמו השתי וערב אשר בשתי שורות שאחת מהן ג' ט"ו כ"ז והשנית ז' ט"ו כ"ג כי כל אחד משני אלו הקטרים ארבעים וחמשה | |style="text-align:right;"|ואם אשר אינם על הקטר הנה כמו השתי וערב אשר בשתי שורות שאחת מהן ג' ט"ו כ"ז והשנית ז' ט"ו כ"ג כי כל אחד משני אלו הקטרים ארבעים וחמשה | ||
|- | |- | ||
| | | | ||
| − | * | + | *The sum of the two extremes of each diagonal is equal to the sum of the two extremes of the corresponding symmetric diagonal. |
| − | |||
|style="text-align:right;"|ונמצא מקובץ שתי קצוות שורה כל שתי וערב שוה למקובץ שתי קצוות השורה האחרת | |style="text-align:right;"|ונמצא מקובץ שתי קצוות שורה כל שתי וערב שוה למקובץ שתי קצוות השורה האחרת | ||
|- | |- | ||
| | | | ||
| − | *the sum of all the cells in | + | *the sum of all the cells in every square equals the 4th power of the number of cells on the side of the square. |
| − | |style="text-align:right;"|ונמצא מקובץ | + | |style="text-align:right;"|ונמצא מקובץ בתי כל מרובע בנוי מאלה המספרים על המשכם שוה למרבע מרבע מספר בתי הצלע |
|- | |- | ||
| | | | ||
| + | ::*For, when one builds a square, whose side is two cells, and these are its numbers: | ||
|style="text-align:right;"|כי אתה כשתבנה מרבע צלעו שני בתים ויהיו מספריו כזה | |style="text-align:right;"|כי אתה כשתבנה מרבע צלעו שני בתים ויהיו מספריו כזה | ||
|- | |- | ||
| | | | ||
| − | {|class="wikitable" style="color: blue; text-align:center;" | + | :::{|class="wikitable" style="color: blue; text-align:center;" |
|- | |- | ||
|3||1 | |3||1 | ||
| Line 804: | Line 882: | ||
|- | |- | ||
| | | | ||
| − | : | + | :::The sum is [<math>\scriptstyle{\color{blue}{1+3+5+7=16=2^4}}</math>] |
|style="text-align:right;"|יהיה מקובץ זה ששה עשר והוא מרבע מרבע שנים | |style="text-align:right;"|יהיה מקובץ זה ששה עשר והוא מרבע מרבע שנים | ||
|- | |- | ||
| | | | ||
| + | ::*And when its sides are of three cells, such as: | ||
|style="text-align:right;"|וכאשר תהיינה צלעותיו משלשה בתים כזה | |style="text-align:right;"|וכאשר תהיינה צלעותיו משלשה בתים כזה | ||
|- | |- | ||
| | | | ||
| − | {|class="wikitable" style="color: blue; text-align:center;" | + | :::{|class="wikitable" style="color: blue; text-align:center;" |
|- | |- | ||
|5||3||1 | |5||3||1 | ||
| Line 832: | Line 911: | ||
|- | |- | ||
| | | | ||
| − | : | + | :::Their sum is: [<math>\scriptstyle{\color{blue}{1+3+5+7+9+11+13+15+17=81=3^4}}</math>] |
|style="text-align:right;"|עד שיהיו מספריו א' ג' ה' ז' ט' י"א י"ג ט"ו י"ז הנה יגיע מקובץ זה לשמנים ואחד והוא מרבע מרבע השלשה | |style="text-align:right;"|עד שיהיו מספריו א' ג' ה' ז' ט' י"א י"ג ט"ו י"ז הנה יגיע מקובץ זה לשמנים ואחד והוא מרבע מרבע השלשה | ||
|- | |- | ||
| | | | ||
| − | + | ::The sum [of the numbers] on the principal diagonal is equal to the cube of the number [of cells on the side of the square]: | |
|style="text-align:right;"|ונמצא הקטר בכל אלו שוה למעקב זה המספר | |style="text-align:right;"|ונמצא הקטר בכל אלו שוה למעקב זה המספר | ||
|- | |- | ||
| | | | ||
| − | : | + | ::*For example, in the greater table, whose [side is] five cells, its principal diagonal is: [<math>\scriptstyle{\color{blue}{1+13+25+37+49=9+17+25+33+41=125=5^3}}</math>] |
|style="text-align:right;"|המשל בזה בלוח הגדול אשר בתיו חמשה הנה קטרו קכ"ה | |style="text-align:right;"|המשל בזה בלוח הגדול אשר בתיו חמשה הנה קטרו קכ"ה | ||
|- | |- | ||
| | | | ||
| − | : | + | ::*On the second [table], the principal diagonal is: [<math>\scriptstyle{\color{blue}{1+7=3+5=8=2^3}}</math>] |
|style="text-align:right;"|ובשני קטרו שמנה | |style="text-align:right;"|ובשני קטרו שמנה | ||
|- | |- | ||
| | | | ||
| − | : | + | ::*On the third [table], the principal diagonal is: [<math>\scriptstyle{\color{blue}{1+9+17=5+9+13=27=3^3}}</math>] |
| − | |style="text-align:right;"|ובשלישי קטרו כ"ז ועל הדרך הזה | + | |style="text-align:right;"|ובשלישי קטרו כ"ז |
| + | |- | ||
| + | | | ||
| + | ::And so on. | ||
| + | |style="text-align:right;"|ועל הדרך הזה | ||
|- | |- | ||
| | | | ||
| + | ::*When one builds a triangular table, as this diagram: | ||
|style="text-align:right;"|והנה כאשר תבנה מהם תמונה משלשת על זאת הצורה | |style="text-align:right;"|והנה כאשר תבנה מהם תמונה משלשת על זאת הצורה | ||
|- | |- | ||
| | | | ||
| − | + | :{|class="wikitable" style="color: blue; text-align:center;" | |
| − | |||
| − | {|style=" | ||
|- | |- | ||
| − | | | + | | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
{| | {| | ||
|- | |- | ||
| − | | | + | | || || || || || ||1|| || || || || || |
| − | |||
| − | | | ||
|- | |- | ||
| − | | | + | | || || || || ||5|| ||3|| || || || || |
| − | |||
| − | | | ||
|- | |- | ||
| − | | | + | | || || || ||11|| ||9|| ||7|| || || || |
| − | |||
| − | | | ||
|- | |- | ||
| − | | | + | | || || ||19|| ||17|| ||15|| ||13|| || || |
| − | |||
| − | | | ||
|- | |- | ||
| − | | | + | | || ||29|| ||27|| ||25|| ||23|| ||21|| || |
| − | |||
| − | |||
| − | |||
| − | | | ||
|- | |- | ||
| − | | | + | | ||41|| ||39|| ||37|| ||35|| ||33|| ||31|| |
| − | | | ||
|- | |- | ||
| + | |55|| ||53|| ||51|| ||49|| ||47|| ||45|| ||43 | ||
| + | |} | ||
| + | |} | ||
| | | | ||
| − | |style=" | + | {|class="wikitable" style="margin-left: auto; margin-right: 0px;" |
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle\sum_{i=1}^n 2i=n^2+n</math> | + | {| |
| − | |style="text-align:right;"| | + | |- |
| + | | || || || || || ||א|| || || || || || | ||
| + | |- | ||
| + | | || || || || ||ה|| ||ג|| || || || || | ||
| + | |- | ||
| + | | || || || ||יא|| ||ט|| ||ז|| || || || | ||
| + | |- | ||
| + | | || || ||יט|| ||יז|| ||טו|| ||יג|| || || | ||
| + | |- | ||
| + | | || ||כט|| ||כז|| ||כה|| ||כג|| ||כא|| || | ||
| + | |- | ||
| + | | ||מא|| ||לט|| ||לז|| ||לה|| ||לג|| ||לא|| | ||
| + | |- | ||
| + | |נה|| ||נג|| ||נא|| ||מט|| ||מז|| ||מה|| ||מג | ||
| + | |} | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | *the numbers on the altitude of the triangle from top to bottom are the successive square numbers | ||
| + | |style="text-align:right;"|תמצא כל המספרים היורדים מן האחד אל נפילת העמוד מרבעים על הסדר | ||
| + | |- | ||
| + | | | ||
| + | *the sum of the numbers in each row breadthwise is a cubic number | ||
| + | |style="text-align:right;"|ותמצא מקובץ מה שבטור אחד ברחב מעקב | ||
| + | |- | ||
| + | | | ||
| + | :::Such as: <math>\scriptstyle{\color{blue}{3+5}}</math>; and: <math>\scriptstyle{\color{blue}{7+9+11}}</math> | ||
| + | |style="text-align:right;"|כמו מקובץ ג' וה' ומקובץ ז' ט' וי"א | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== Even Number ==== | ||
| + | |||
| + | |style="text-align:right;"|<big>ואמנם</big> מספר הזוג | ||
| + | |- | ||
| + | |Whatever is known concerning to it, is known in the book of the ''Elements''. | ||
| + | |style="text-align:right;"|כבר ידעת בספר היסודות ממנו מה שידעת | ||
| + | |- | ||
| + | |Properties that are necessary to its terms will be referred to: | ||
| + | |style="text-align:right;"|ונרמוז לך אל סגלות יתחיבו למדרגותיו | ||
| + | |- | ||
| + | | | ||
| + | *Among them: the sum of its terms is equal to the square of the number of the terms plus its side. | ||
| + | :<math>\scriptstyle\sum_{i=1}^n 2i=n^2+n</math> | ||
| + | |style="text-align:right;"|מהן שאתה תמצא מקובץ מדרגותיו שוה למרבע מספר המדרגות מצורף אליו צלעו | ||
|- | |- | ||
| | | | ||
| − | : | + | ::Such as: <math>\scriptstyle{\color{blue}{2+4=\sum_{i=1}^2 2i=6=2^2+2}}</math> |
|style="text-align:right;"|כמו שאתה כאשר תתחיל מהשנים ותצרף אליהם הארבעה יהיו ששה והוא כמו מרבע מספר המדרגות שהם שנים וכמו צלעו | |style="text-align:right;"|כמו שאתה כאשר תתחיל מהשנים ותצרף אליהם הארבעה יהיו ששה והוא כמו מרבע מספר המדרגות שהם שנים וכמו צלעו | ||
|- | |- | ||
| | | | ||
| − | : | + | ::And as: <math>\scriptstyle{\color{blue}{2+4+6=\sum_{i=1}^3 2i=12=3^2+3}}</math> |
|style="text-align:right;"|וכמו שאתה כאשר תתחיל מהשנים ותצרף אליהם הארבעה והששה יהיו שנים עשר והוא כמו מרבע השלשה וכמו צלעו | |style="text-align:right;"|וכמו שאתה כאשר תתחיל מהשנים ותצרף אליהם הארבעה והששה יהיו שנים עשר והוא כמו מרבע השלשה וכמו צלעו | ||
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle2m=p+1=\left(\frac{1}{p}\sdot p^2\right)+\left(\frac{1}{p^2}\sdot p^2\right)</math> | + | *Every even number that exceeds a prime odd number by one, is equal to the sum of the divisors of the square of this prime number. |
| − | |style="text-align:right;"|ומסגלותיו שכל זוג יוסיף על הראשון מהנפרדים באחד הנה זה הזוג שוה למקובץ חלקי מרבע זה הראשון | + | :<math>\scriptstyle2m=p+1=\left(\frac{1}{p}\sdot p^2\right)+\left(\frac{1}{p^2}\sdot p^2\right)</math> |
| + | |style="text-align:right;"|<big>ומסגלותיו</big> שכל זוג יוסיף על הראשון מהנפרדים באחד הנה זה הזוג שוה למקובץ חלקי מרבע זה הראשון | ||
|- | |- | ||
| | | | ||
| − | : | + | ::Such as 4: <math>\scriptstyle{\color{blue}{4=3+1=\left(\frac{1}{3}\sdot9\right)+\left(\frac{1}{9}\sdot9\right)=\left(\frac{1}{3}\sdot3^2\right)+\left(\frac{1}{3^2}\sdot3^2\right)}}</math> |
|style="text-align:right;"|כמו הארבעה אשר הוא מוסיף על השלשה שהוא ראשון באחד ומרבע השלשה תשעה ולהם מהחלקים שני חלקים תשיעית ושלישית ומקובצם שוה אל הארבעה | |style="text-align:right;"|כמו הארבעה אשר הוא מוסיף על השלשה שהוא ראשון באחד ומרבע השלשה תשעה ולהם מהחלקים שני חלקים תשיעית ושלישית ומקובצם שוה אל הארבעה | ||
|- | |- | ||
| | | | ||
| − | : | + | ::And also 6: <math>\scriptstyle{\color{blue}{6=5+1=\left(\frac{1}{5}\sdot25\right)+\left(\frac{1}{25}\sdot25\right)=\left(\frac{1}{5}\sdot5^2\right)+\left(\frac{1}{5^2}\sdot5^2\right)}}</math> |
|style="text-align:right;"|וג"כ הששה יוסיף באחד על החמשה שהוא נפרד ראשון ומרבע זה הראשון עשרים וחמשה ולו מן החלקים חומש וחומש החומש לא זולתם והגעתם ששה | |style="text-align:right;"|וג"כ הששה יוסיף באחד על החמשה שהוא נפרד ראשון ומרבע זה הראשון עשרים וחמשה ולו מן החלקים חומש וחומש החומש לא זולתם והגעתם ששה | ||
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle2m=p+3=\left(\frac{1}{2}\sdot2p\right)+\left(\frac{1}{p}\sdot2p\right)+\left(\frac{1}{2p}\sdot2p\right)</math> | + | *For an even number, such that when 3 is subtracted from it, the remainder is a prime odd number, this even number consists of the divisors of double this odd number. |
| + | :<math>\scriptstyle2m=p+3=\left(\frac{1}{2}\sdot2p\right)+\left(\frac{1}{p}\sdot2p\right)+\left(\frac{1}{2p}\sdot2p\right)</math> | ||
|style="text-align:right;"|ואמנם אם יהיה הזוג באופן שכאשר נגרע ממנו שלשה ישאר נפרד ראשון הנה זה הזוג מורכב מחלקי כפל זה הנפרד | |style="text-align:right;"|ואמנם אם יהיה הזוג באופן שכאשר נגרע ממנו שלשה ישאר נפרד ראשון הנה זה הזוג מורכב מחלקי כפל זה הנפרד | ||
|- | |- | ||
| | | | ||
| − | : | + | ::Such as: 8 |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כמו השמנה |
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{2\sdot\left(8-3\right)=2\sdot5=10}}</math> | ||
| + | |style="text-align:right;"|שהם כאשר נגרע מהם שלשה נשארו חמשה וכפלם עשרה | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(\frac{1}{2}\sdot10\right)+\left(\frac{1}{5}\sdot10\right)+\left(\frac{1}{10}\sdot10\right)=5+2+1=8}}</math> | ||
| + | |style="text-align:right;"|ולהם חצי וחומש ועשירית ומקובץ זה שמנה רצוני מקובץ החמשה והשנים והאחד | ||
|} | |} | ||
{| | {| | ||
| Line 949: | Line 1,054: | ||
==== Types of Even and Odd Numbers ==== | ==== Types of Even and Odd Numbers ==== | ||
| − | |style="text-align:right;"|ונדבר | + | |style="text-align:right;"|<big>ונדבר</big> עתה בסגלות מיני הזוג ומיני הנפרד |
|- | |- | ||
| − | | | + | |Starting from the properties of the even number, as their categorization to types is more reasonable than the categorization of the odd numbers. |
| − | |style="text-align:right;"|ונתחיל בסגלות | + | |style="text-align:right;"|ונתחיל בסגלות ה{{#annot:term|63,1333|wfI7}}זוג{{#annotend:wfI7}} לפי שחלוקתם אל מינים יותר קרובה מחלוקת הנפרדים |
|- | |- | ||
| | | | ||
| Line 958: | Line 1,063: | ||
| | | | ||
| + | |- | ||
| + | |Starting from the properties of the even-times-even number, since it is simpler. | ||
| + | |style="text-align:right;"|ונתחיל בסגלות הזוג הזוג לפי שהוא יותר פשוט | ||
|- | |- | ||
| | | | ||
====== Even-Times Even ====== | ====== Even-Times Even ====== | ||
| − | | | + | | |
|- | |- | ||
| − | | | + | |The quality of its production by way of doubling and other of its properties that are in the book of the ''Elements'', are already known. |
|style="text-align:right;"|וכבר ידעת איכות התילדותו על דרך ההכפלה וסגלות אחרות מהסגלות אשר לו בספר היסודות‫<ref>marg. בל"ד מט'</ref> | |style="text-align:right;"|וכבר ידעת איכות התילדותו על דרך ההכפלה וסגלות אחרות מהסגלות אשר לו בספר היסודות‫<ref>marg. בל"ד מט'</ref> | ||
|- | |- | ||
| − | | | + | |Of the properties of the even-times-even number there are parts of properties that were mentioned in the ''Elements''. |
| − | |style="text-align:right;"|והנה מסגלות זוג הזוג מה שהם סעיפים מסגלות נזכרו ביסודות | + | |style="text-align:right;"|<ref>והנה</ref> מסגלות {{#annot:term|1334,69|a7rS}}זוג הזוג{{#annotend:a7rS}} מה שהם סעיפים מסגלות נזכרו ביסודות |
|- | |- | ||
| | | | ||
| − | *not divisible by an odd number | + | *It has no part that is denominated by [= it is not divisible by] an odd number, or an even number that is not an even-times-even-number |
| − | |style="text-align:right;"|שהוא אין חלק לו נקרא בשם מספר נפרד | + | |style="text-align:right;"|שהוא אין חלק לו נקרא בשם {{#annot:term|65,1336|8fTS}}מספר נפרד{{#annotend:8fTS}} ולא זוג בלתי זוג הזוג‫<ref>marg. כי לפי זה יחויב שיהיו השנים נמנים במדרגות זוג הזוג</ref> |
|- | |- | ||
| | | | ||
| − | * | + | *There is no smaller even-times-even number that does not divide it. |
| − | |||
| − | |||
| − | |||
| − | |||
|style="text-align:right;"|ואין זוג הזוג שהוא פחות ממנו שלא ימנה אותו | |style="text-align:right;"|ואין זוג הזוג שהוא פחות ממנו שלא ימנה אותו | ||
|- | |- | ||
| | | | ||
| − | *its square is even-times-even number | + | *For every even-times-even number, its square is and even-times-even number. |
|style="text-align:right;"|וכל זוג זוג הנה מרבעו זוג הזוג | |style="text-align:right;"|וכל זוג זוג הנה מרבעו זוג הזוג | ||
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle2^n-2=2\sdot\left(2^{n-1}-1\right)</math> → even-times-odd number | + | *When the first even number, which is two, is subtracted from it, the result is an even-times-odd number. |
| − | |style="text-align:right;"|וכאשר חוסר ממנו הזוג הראשון והוא שנים יצא זוג הנפרד‫<ref>marg. א"ב לפי זה יהיו השנים זוג הנפרד וזה כי הארבעה זוג הזוג וכאשר נגרע מהם הזוג הראשון שהוא שנים ישארו שנים ואם שנים זוג הנפרד המאמר צודק ולא בזולת זה ובזה תמה כי במקומות מהספר נראה שהוא לוקח השנים | + | :<math>\scriptstyle2^n-2=2\sdot\left(2^{n-1}-1\right)</math> → even-times-odd number |
| + | |style="text-align:right;"|וכאשר חוסר ממנו הזוג הראשון והוא שנים יצא זוג הנפרד‫<ref>marg. א"ב לפי זה יהיו השנים זוג הנפרד וזה כי הארבעה זוג הזוג וכאשר נגרע מהם הזוג הראשון שהוא שנים ישארו שנים ואם שנים זוג הנפרד המאמר צודק ולא בזולת זה ובזה תמה כי במקומות מהספר נראה שהוא לוקח השנים ב{{#annot:term|1334,69|wsFA}}מספר זוג הזוג{{#annotend:wsFA}} ובמקומות ב{{#annot:term|70,2125|17FM}}מספר זוג הנפרד{{#annotend:17FM}}</ref> | ||
|- | |- | ||
| | | | ||
| − | : | + | ::Such as 8: <math>\scriptstyle{\color{blue}{8-2=2^3-2=2\sdot3=6}}</math> |
|style="text-align:right;"|כמו השמנה שנחסר מהם שנים ויצא זוג הנפרד והוא ששה | |style="text-align:right;"|כמו השמנה שנחסר מהם שנים ויצא זוג הנפרד והוא ששה | ||
|- | |- | ||
| | | | ||
| − | * | + | *Every even-times-even is deficient, and its deficiency is one [= the sum of its parts is the number minus 1. |
| + | :<math>\scriptstyle\sum_{i=1}^n 2^{i-1}=2^n-1</math>] | ||
|style="text-align:right;"|וכל זוג הזוג הנה הוא חסר וחסרונו באחד‫<ref>marg. פי' כמו שמנה שהוא זוג הזוג וחלקיו שהם חצי ורביע ושמינית והם ד'ב'א' והגעתם שבעה</ref> | |style="text-align:right;"|וכל זוג הזוג הנה הוא חסר וחסרונו באחד‫<ref>marg. פי' כמו שמנה שהוא זוג הזוג וחלקיו שהם חצי ורביע ושמינית והם ד'ב'א' והגעתם שבעה</ref> | ||
|- | |- | ||
| | | | ||
| − | * | + | *Among the properties of the even-times-even number: its terms follow by the geometric proportion. |
| − | |style="text-align:right;"|ומסגלות זוג הזוג שמדרגותיו נמשכות על יחס מתדמה תשברתיי‫<ref>marg. ירצה לפי שיש ג' מיני יחסי' יחס מדותיי והוא שהשעורי' מתיחסי' והמותרי' בלתי שוי' ויחס מספרי והוא שהשעורי' בלתי מתיחסי' והמותרי' שוים יחס מוסיף והוא שהשעורי' בלתי מתיחסי' והמותרי' בלתי שוי' אבל יחס השעור הא' אל הג' כיחס מותר מה שבין השעור הא' אל הב' אל מותר מה שבין השעור הב' אל השעו' השלישי כמו מספרי' ג'ד'ו' א"ב ר"ל</ref> | + | :<math>\scriptstyle2^n:2^{n-1}=2^{n+1}:2^{n}</math> |
| + | |style="text-align:right;"|<big>ומסגלות</big> זוג הזוג שמדרגותיו נמשכות על יחס מתדמה תשברתיי‫<ref>marg. ירצה לפי שיש ג' מיני יחסי' יחס מדותיי והוא שהשעורי' מתיחסי' והמותרי' בלתי שוי' ויחס מספרי והוא שהשעורי' בלתי מתיחסי' והמותרי' שוים יחס מוסיף והוא שהשעורי' בלתי מתיחסי' והמותרי' בלתי שוי' אבל יחס השעור הא' אל הג' כיחס מותר מה שבין השעור הא' אל הב' אל מותר מה שבין השעור הב' אל השעו' השלישי כמו מספרי' ג'ד'ו' א"ב ר"ל</ref> | ||
|- | |- | ||
| | | | ||
| − | + | :Since they follow by the doubling way. | |
|style="text-align:right;"|לפי שהם נמשכות על דרך ההכפל | |style="text-align:right;"|לפי שהם נמשכות על דרך ההכפל | ||
|- | |- | ||
| | | | ||
| − | + | :And their excesses are unequal. | |
| + | :<math>\scriptstyle2^n-2^{n-1}\ne2^{n+1}-2^n</math> | ||
|style="text-align:right;"|והנה לא תהיינה העדפותיהם שוות | |style="text-align:right;"|והנה לא תהיינה העדפותיהם שוות | ||
|- | |- | ||
| | | | ||
| − | + | :But, each excess is equal to that over which it exceeds. | |
| + | :<math>\scriptstyle2^n-2^{n-1}=2^{n-1}</math> | ||
|style="text-align:right;"|אבל יהיה כל העדף שוה למועדף עליו | |style="text-align:right;"|אבל יהיה כל העדף שוה למועדף עליו | ||
|- | |- | ||
| | | | ||
| − | + | :The difference between the excesses is the excess itself. | |
| + | :<math>\scriptstyle\left(2^{n+1}-2^n\right)-\left(2^n-2^{n-1}\right)=2^n-2^{n-1}</math> | ||
|style="text-align:right;"|ותהיינה ההעדפות עודפות בזה שביניהם זה ההעדף בעצמו | |style="text-align:right;"|ותהיינה ההעדפות עודפות בזה שביניהם זה ההעדף בעצמו | ||
|- | |- | ||
| | | | ||
| + | *It follows necessarily from its terms being in one ratio, that they are proportional when they are divided and when they are multiplied equally. | ||
|style="text-align:right;"|ויתחיב מהיות מדרגותיו על היחס האחד שתהיינה מתיחסות כשנחתוך אותם ומתיחסות כשנוסיף עליהם על השווי‫<ref>marg. ירצה כשנקח מהם חלקי' מתיחסי' על יחס א' הן לתוספת הן למגרעת כמו עד"מ שנקח החצי או הרביע מכל א' מהם או כפל או ג' כפלי כל א' מהם ודומה לזה וישמרו תמיד יחס הכפל עצמו.. א"ב ר"ל</ref> | |style="text-align:right;"|ויתחיב מהיות מדרגותיו על היחס האחד שתהיינה מתיחסות כשנחתוך אותם ומתיחסות כשנוסיף עליהם על השווי‫<ref>marg. ירצה כשנקח מהם חלקי' מתיחסי' על יחס א' הן לתוספת הן למגרעת כמו עד"מ שנקח החצי או הרביע מכל א' מהם או כפל או ג' כפלי כל א' מהם ודומה לזה וישמרו תמיד יחס הכפל עצמו.. א"ב ר"ל</ref> | ||
|- | |- | ||
| | | | ||
| − | *the | + | *It follows necessarily that the product of whichever mean by itself is as the product of one of the two sides by the other. |
| + | :<math>\scriptstyle\left(2^n\right)^2=2^{n-m}\sdot2^{n+m}</math> | ||
|style="text-align:right;"|ויתחיב שיהיה הכאת אי זה אמצעי שנקח בעצמו כהכאת אחת מהשתי פאות באחרת | |style="text-align:right;"|ויתחיב שיהיה הכאת אי זה אמצעי שנקח בעצמו כהכאת אחת מהשתי פאות באחרת | ||
|- | |- | ||
| | | | ||
| − | + | :As the ratio of the smaller side to the mean is as the ratio of the mean to the greater side. | |
| − | |style="text-align:right;"|לפי שיחס | + | :<math>\scriptstyle2^{n-m}:2^n=2^n:2^{n+m}</math> |
| + | |style="text-align:right;"|לפי שיחס ה{{#annot:term|1465,327|wYlv}}פאה{{#annotend:wYlv}} הקטנה אל האמצעי כיחס האמצעי אל הפאה הגדולה | ||
|- | |- | ||
| | | | ||
| − | *the product of the two means is | + | *It follows necessarily that the product of one of the two means by the other is as the product of one of the two sides by the other. |
| + | :<math>\scriptstyle2^n\sdot2^{n+1}=2^{n-m}\sdot2^{n+1+m}</math> | ||
|style="text-align:right;"|ויתחיב שתהיה הכאת אחד מהשני אמצעיים באחר כהכאת אחת מהשתי פאות באחרת | |style="text-align:right;"|ויתחיב שתהיה הכאת אחד מהשני אמצעיים באחר כהכאת אחת מהשתי פאות באחרת | ||
|- | |- | ||
| | | | ||
| − | + | :As the ratio of the smaller side to the smaller mean is as the ratio of the greater mean to the greater side. | |
| + | :<math>\scriptstyle2^{n-m}:2^n=2^{n+1}:2^{n+1+m}</math> | ||
|style="text-align:right;"|לפי שיחס הפאה הקטנה אל האמצעי הקטן כיחס האמצעי הגדול אל הפאה הגדולה | |style="text-align:right;"|לפי שיחס הפאה הקטנה אל האמצעי הקטן כיחס האמצעי הגדול אל הפאה הגדולה | ||
|- | |- | ||
| | | | ||
| − | : | + | ::Such as the terms: <span style="color:#0000FF>4; 8; 16; 32; 64</span> |
| − | + | |style="text-align:right;"|והנה תהיינה המדרגות ב' ד' ח' י"ו ל"ב ס"ד | |
| − | |||
| − | |||
| − | |||
| − | |style="text-align:right;"|והנה תהיינה המדרגות ב' ד' ח' י"ו ל"ב ס | ||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | + | :::<math>\scriptstyle{\color{blue}{4^2=2\sdot8}}</math> | |
| − | + | |style="text-align:right;"|ונמצא ד' בעצמו כמו ב' בח‫' | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | :::<math>\scriptstyle{\color{blue}{8^2=2\sdot32=4\sdot16}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וח' בעצמו כמו ב' בל"ב וד' בי"ו |
|- | |- | ||
| | | | ||
| − | + | :::<math>\scriptstyle{\color{blue}{4\sdot8=2\sdot16}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ונמצא ד' בח' כמו ב' בי"ו |
|- | |- | ||
| | | | ||
| − | : | + | :::<math>\scriptstyle{\color{blue}{8\sdot16=4\sdot32=2\sdot64}}</math> |
| − | ::<math>\scriptstyle{\color{blue}{\ | + | |style="text-align:right;"|וח' בי"ו כמו ד' בל"ב וב' בס"ד |
| − | |style="text-align:right;"|כמו | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | ::{|style="color: blue;" | + | *Since the even-times-even numbers are arranged by a continuous ratio, it follows necessarily that the squares and the cubes among them have sequences, as the third from a square is a square, and the fourth from a cube is a cube. |
| − | |142 | + | :<math>\scriptstyle a_n=2^{2m}\longrightarrow a_{n+2}=2^{2\sdot\left(m+1\right)}</math> |
| + | :<math>\scriptstyle a_n=2^{3m}\longrightarrow a_{n+3}=2^{3\sdot\left(m+1\right)}</math> | ||
| + | |style="text-align:right;"|ולפי שהיו {{#annot:term|1334,69|kOjL}}מספרי זוג הזוג{{#annotend:kOjL}} מסודרים על יחס מתדבק יחויב שיהיה למרבעים ולמעקבים מהם סדר באשר המרבע יהיה השלישי אליו מרבע והמעקב הרביעי אליו מעקב וילך על הדרך הזה | ||
| + | |- | ||
| + | | | ||
| + | *Among its properties: the '''perfect numbers''' are generated from it. | ||
| + | |style="text-align:right;"|<big>ומסגלותיו</big> שהמספרים השלמים יתילדו ממנו‫<ref>marg. מל"ה מט'</ref> | ||
| + | |- | ||
| + | | | ||
| + | *The '''amicable numbers''' are the numbers that are compounded each from the parts of its amicable number, as the amicable number is compounded from its own parts. | ||
| + | |style="text-align:right;"|<big>אמנם</big> המספרים הנאהבים הנה הם המספרים אשר יתרכבו כל אחד מחלקי חברו כמו שיתרכב חברו מחלקיו | ||
| + | |- | ||
| + | | | ||
| + | ::Such as: <span style="color:#0000FF>220; 284</span> | ||
| + | |style="text-align:right;"|כמו מאתים ועשרים ממאתים ושמנים וארבעה | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{\frac{1}{2}\sdot284=142}}</math> | ||
| + | |style="text-align:right;"|כי למאתים ושמנים וארבעה חצי והוא קמ"ב | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{\frac{1}{4}\sdot284=71}}</math> | ||
| + | |style="text-align:right;"|להם רביעית והוא ע"א | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{\frac{1}{71}\sdot284=4}}</math> | ||
| + | |style="text-align:right;"|ולהם חלק מאחד ושבעים והוא ד‫' | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{\frac{1}{142}\sdot284=2}}</math> | ||
| + | |style="text-align:right;"|ולהם חלק ממאה וארבעים ושנים והוא ב‫' | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{\frac{1}{284}\sdot284=1}}</math> | ||
| + | |style="text-align:right;"|ולהם חלק ממאתים ושמנים וארבעה והוא א‫' | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{142+71+4+2+1=220}}</math> | ||
| + | |style="text-align:right;"|ואומר נקבץ אלה החלקים יהיו ר"כ | ||
| + | |- | ||
| + | | | ||
| + | ::{|style="color: blue;" | ||
| + | |142 | ||
|- | |- | ||
| 71 | | 71 | ||
| Line 1,104: | Line 1,245: | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{ | + | :::<math>\scriptstyle{\color{blue}{\frac{1}{2}\sdot220=110}}</math> |
| − | |style="text-align:right;"|ואמנם חלקי ר"כ הנה להם החצי והוא ק"י | + | |style="text-align:right;"|ואמנם חלקי ר"כ הנה להם החצי והוא ק"י |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | + | :::<math>\scriptstyle{\color{blue}{\frac{1}{4}\sdot220=55}}</math> | |
| − | + | |style="text-align:right;"|ורביעית והוא נ"ה | |
| − | |||
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | :::<math>\scriptstyle{\color{blue}{\frac{1}{5}\sdot220=44}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וחמישית והוא מ"ד |
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | + | :::<math>\scriptstyle{\color{blue}{\frac{1}{10}\sdot220=22}}</math> | |
| − | = | + | |style="text-align:right;"|ועשירית והוא כ"ב |
| − | |||
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :::<math>\scriptstyle{\color{blue}{\frac{1}{11}\sdot220=20}}</math> |
| + | |style="text-align:right;"|וחלק מאחד עשר והוא עשרים | ||
|- | |- | ||
| | | | ||
| − | + | :::<math>\scriptstyle{\color{blue}{\frac{1}{20}\sdot220=11}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וחלק מעשרים והוא י"א |
|- | |- | ||
| | | | ||
| − | + | :::<math>\scriptstyle{\color{blue}{\frac{1}{22}\sdot220=10}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וחלק מכ"ב והוא עשרה |
|- | |- | ||
| | | | ||
| − | : | + | :::<math>\scriptstyle{\color{blue}{\frac{1}{44}\sdot220=5}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וחלק ממ"ד והוא ה‫' |
|- | |- | ||
| | | | ||
| − | + | :::<math>\scriptstyle{\color{blue}{\frac{1}{55}\sdot220=4}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וחלק מנ"ה והוא ד‫' |
|- | |- | ||
| | | | ||
| − | : | + | :::<math>\scriptstyle{\color{blue}{\frac{1}{110}\sdot220=2}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וחלק מק"י והוא ב‫' |
|- | |- | ||
| | | | ||
| − | + | :::<math>\scriptstyle{\color{blue}{\frac{1}{220}\sdot220=1}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וחלק מר"כ והוא א‫' |
|- | |- | ||
| | | | ||
| − | + | ::<math>\scriptstyle{\color{blue}{110+55+44+22+20+11+10+5+4+2+1=284}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכאשר תקבץ אלה החלקים יהיו רפ"ד |
|- | |- | ||
| | | | ||
| − | + | ::None of them has parts other than those that were mentioned. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואין לאחד מהם מן החלקים זולת מה שזכרנו |
|- | |- | ||
| | | | ||
| − | + | :Their production technique: | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואופן התילדותם |
|- | |- | ||
| | | | ||
| − | * | + | :*Summing the even-times-even numbers together with 1, so that the sum is a prime number, and when the last term is added to it or subtracted from it, the result of the addition [<math>\scriptstyle\left(\sum_{i=1}^n 2^{i-1}\right)+2^{n-1}</math>] and the result of the subtraction [<math>\scriptstyle\left(\sum_{i=1}^n 2^{i-1}\right)-2^{n-1}</math>] are prime numbers. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|הוא כאשר תקבץ זוג הזוג והאחד עמהם ויקובץ מספר ראשון בתנאי שיהיה כאשר יתוסף עליו האחרון שבהם או יחוסר ממנו אשר לפניו יהיה המגיע אחר התוספת והמגיע אחר החסרון מספר ראשון |
|- | |- | ||
| | | | ||
| − | : | + | :*The product of the result of addition by the result of subtraction, multiplied by the last added term, is a number that has an amicable number: |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle a=\left[\left(\sum_{i=1}^n 2^{i-1}\right)+2^{n-1}\right]\sdot\left[\left(\sum_{i=1}^n 2^{i-1}\right)-2^{n-1}\right]\sdot2^{n-1}</math> |
| + | |style="text-align:right;"|הנה הכאת המגיע הנוסף עליו במגיע המחוסר אחר כן הכאת העולה באחרון שבמקובצים הוא מספר אשר לו אוהב | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*Its amicable number is the number that resulted from adding the product of the sum of the mentioned result of addition and the result of subtraction, multiplied by the last of the summed terms, to the number that was found first, which is the amicable number, and they are amicable numbers. |
| + | ::<math>\begin{align}\scriptstyle b&\scriptstyle=\left[\left[\left(\sum_{i=1}^n 2^{i-1}\right)+2^{n-1}\right]\sdot\left[\left(\sum_{i=1}^n 2^{i-1}\right)-2^{n-1}\right]\sdot2^{n-1}\right]\\&\scriptstyle+\left[\left[\left[\left(\sum_{i=1}^n 2^{i-1}\right)+2^{n-1}\right]+\left[\left(\sum_{i=1}^n 2^{i-1}\right)-2^{n-1}\right]\right]\sdot2^{n-1}\right]\\\end{align}</math> | ||
| + | |style="text-align:right;"|ואוהבו הוא המספר אשר יהיה מתוספת הכאת מקובץ הנוסף והמחוסר הנזכרים באחרון שבמקובצים על המספר הנמצא ראשונה אשר הוא אוהב והם נאהבים‫<ref>marg. א"ב כדי זה המשפט להביא בו משל והנה נקבץ {{#annot:term|1334,69|fHMZ}}זוגי הזוג{{#annotend:fHMZ}} מספרי ב' ד' והאחד עמהם ויהיו ז' והוא מספר ראשון ונוסיף עליו אחרון המקובצי' והוא ד' ויהיו י"א וגם הוא ראשון ונחסר ממנו ר"ל מז' המספר אשר לפני אחרון המקובצים והוא ב' וישאר ה' וגם הוא ראשון ונכה י"א והוא הנוסף בה' שהוא המחוסר ויעלה נ"ה הנה הכאת נ"ה באחרון המקובצים והוא ד' יביא ר"ך והוא המספר אשר לו אוהב והוצאת אוהבו שנוסיף הכאת מקובץ הנוסף והחסר הנזכרי' והוא י"ו בד' שהוא אחרון המקובצים שיעלה ס"ד על ר"ך שהוא האוהב הראשון ויהיה רפ"ד שהוא האוהב השני ושניהם נאהבים ועל הדרך הזה</ref> | ||
| + | |} | ||
| + | {| | ||
|- | |- | ||
| | | | ||
| − | + | ||
| − | + | ====== Even-Times Odd ====== | |
| − | + | ||
| − | : | + | |style="text-align:right;"|<big>ואמנם</big> סגלות {{#annot:term|70,2125|6Juj}}זוג הנפרד{{#annotend:6Juj}} |
| − | + | |- | |
| − | + | |Whatever is known concerning to it, is known in the book of the ''Elements''. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|הנה כבר נודע בספר היסודות ממנו מה שנודע |
| − | + | |- | |
| − | + | |It is clear from their rule: | |
| − | + | |style="text-align:right;"|ונתבאר מכללם | |
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | * | + | *It is divided by an even number only with an odd number, and by an odd number only with an even number. |
| − | |style="text-align:right;"| | + | :[even-times-odd ÷ even = odd; even-times-odd ÷ odd = even] |
| + | |style="text-align:right;"|שהוא לא ימנהו זוג אלא במספר נפרד ולא נפרד אלא ב{{#annot:term|63,1333|AGaI}}מספר זוג{{#annotend:AGaI}} | ||
|- | |- | ||
| | | | ||
| − | * | + | *Its even part is denominated by a name of an odd number. |
| − | + | |style="text-align:right;"|וחלקו הזוג נקרא בשם הנפרד | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | ::Such as: <math>\scriptstyle{\color{blue}{\frac{1}{3}\sdot6=2}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כמו השנים שהם שלישית הששה |
|- | |- | ||
| | | | ||
| − | * | + | *Its odd part is denominated by a name of an even number. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וחלקו הנפרד נקרא בשם הזוג |
|- | |- | ||
| | | | ||
| − | + | ::Such as: <math>\scriptstyle{\color{blue}{\frac{1}{2}\sdot6=3}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כמו השלשה שהוא חצי הששה |
|- | |- | ||
| | | | ||
| − | + | *When the first even number, which is 2, is added to it, it yields an even-times-even[-times-odd] number. | |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle\left[2\sdot\left(2n-1\right)\right]+2=2\sdot2n</math> |
| + | |style="text-align:right;"|ושתוספת הזוג הראשון והוא השנים עליו יוציא זוג הזוג‫<ref>marg. ר"ל שיחלק יותר מפעם אחת לא זוג הזוג שנאמר ביחוד על המתחלק תמיד עד האחד</ref> | ||
|- | |- | ||
| | | | ||
| − | + | *Know that its production is from the products of the successive odd numbers by 2. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ודע שהתילדותו מכפל הנפרדים הנמשכים בשנים |
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle | + | *The difference between each term and the successive term is double the difference between the natural odd numbers. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle\left[2\sdot\left[\left[2\sdot\left(n+1\right)\right]-1\right]\right]-\left[2\sdot\left(2n-1\right)\right]=2\sdot\left[\left[\left[2\sdot\left(n+1\right)\right]-1\right]-\left(2n-1\right)\right]</math> |
| + | |style="text-align:right;"|והנה תדע מזה שהנופל בין מדרגה ובין המדרגה הנמשכת אליה כפל הנופל אשר היה בנפרדים הטבעיים | ||
|- | |- | ||
| | | | ||
| − | + | *The excess of its terms is four. | |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle\left[2\sdot\left[\left[2\sdot\left(n+1\right)\right]-1\right]\right]-\left[2\sdot\left(2n-1\right)\right]=4</math> |
| + | |style="text-align:right;"|ויהיה העדף מדרגותיו בארבעה ארבעה | ||
|- | |- | ||
| | | | ||
| − | + | *There is none that has a square root among them, nor a cube. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ושהוא אין נגדר בו ולא מעקב |
|- | |- | ||
| | | | ||
| − | + | :Since, for every one that has a square root and every cube: if it is an odd number, it is divided by an odd number with an odd number; if it is an even number, it is divided by an even number with an even number. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כי כל נגדר וכל מעקב אם נפרד ימנה בנפרד במספר נפרד ואם זוג ימנה בזוג במספר זוג |
|- | |- | ||
| | | | ||
| − | : | + | :This is already known, as the excess is four. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכבר ידעת זה לפי שהיה ההעדף בארבעה ארבעה |
|- | |- | ||
| | | | ||
| − | + | ::The beginning either from 2, or from 6, as the property will be explained. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וההתחלה אם מהשנים ואם מהששה כפי מה שיתבאר הענין בו |
|- | |- | ||
| | | | ||
| − | + | ::<math>\scriptstyle{\color{blue}{2+4=6}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והשנים כאשר נוסיף עליהם ארבעה יהיו ששה |
|- | |- | ||
| | | | ||
| − | : | + | ::<math>\scriptstyle{\color{blue}{6+4=10}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכאשר נוסיף על הששה ארבעה יהיו עשרה |
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{10+4=14}}</math> |
| + | |style="text-align:right;"|וכאשר נוסיף עליהם ארבעה יהיו ארבעה עשר | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{14+4=18}}</math> |
| + | |style="text-align:right;"|וכאשר נוסיף עליהם ארבעה יהיו שמנה עשר | ||
|- | |- | ||
| | | | ||
| − | {| | + | ::<math>\scriptstyle{\color{blue}{18+4=22}}</math> |
| + | |style="text-align:right;"|וכאשר נוסיף עליהם ארבעה יהיו שנים ועשרים | ||
|- | |- | ||
| − | | | + | | |
| + | ::Hence, returning to 2, recurring cyclically. | ||
| + | |style="text-align:right;"|והנה שבנו אל השנים חוזרים בסבוב | ||
|- | |- | ||
| − | | | + | | |
| + | *It follows necessarily that there is one cycle that repeats in the others and their units are similar to the units of the first cycle [i.e. 2, 6, 0, 4, 8] | ||
| + | |style="text-align:right;"|יחויב שיהיה סבוב אחד האחרים זולת אלו ויחויב שיהיה כל דומה אל הראשון באחדים או גלגל הנקרא {{#annot:term|1813,205|TfGB}}ציפרי{{#annotend:TfGB}} | ||
|- | |- | ||
| − | | | + | | |
| + | *When starting from <span style="color: blue;">6</span>, which has a third [= divisible by 3] that is denominated by three, the third successive term after it, which is <span style="color: blue;">18</span>, has a third [= divisible by 3], and the third successive term after 18, which is <span style="color: blue;">30</span>, has a third, and so on endlessly. | ||
| + | :[→ <math>\scriptstyle6+\left(3\sdot4n\right)</math> are even-times-odd numbers divisible by 3] | ||
| + | |style="text-align:right;"|וכאשר תשים התחלת המדרגות מהששה והששה להם שליש והם הנקראים על שם שלשה הנה כאשר תתחיל אחר הששה תמצא לשלישי אחריו והוא שמנה עשר שליש אמתי ולשלישי כאשר תתחיל אחר השמנה עשר והוא השלשים שליש אמתי ועל הדרך הזה אל בלתי תכלית | ||
|- | |- | ||
| − | | | + | | |
| + | *The successive term after 6 is 10 and its part is denominated by the successive odd after 3, which is 5, for 10 has a fifth [= divisible by 5], hence, when starting from <span style="color: blue;">10</span>, the fifth successive term after it has a fifth [= divisible by 5], and so on as much as one wishes. | ||
| + | :[→ <math>\scriptstyle10+\left(5\sdot4n\right)</math> are even-times-odd numbers divisible by 5] | ||
| + | |style="text-align:right;"|ואחר הששה העשרה והחלק שלהם נקרא בשם הנפרד אשר אחר השלשה והוא החמשה כי לעשרה חומש אמתי והנה כאשר תתחיל אחר העשרה תמצא הנגזר לו השם מזה המספר והוא החמישי לו חומש אמתי ועל הדרך הזה עד מה שתרצה | ||
|- | |- | ||
| − | | | + | | |
| + | *The successive term after 10 is 14 and its part is denominated by the successive odd after 5, for it has a seventh [= divisible by 7], hence, the seventh successive term after it is found [divisible by 7], and so on. | ||
| + | :[→ <math>\scriptstyle14+\left(7\sdot4n\right)</math> are even-times-odd numbers divisible by 7] | ||
| + | |style="text-align:right;"|והמספר אשר אחר העשרה הוא הארבעה עשר וחלקו נקרא בשם הנפרד הנמשך אל החמשה כי הנה לו שביעית והנה נמצא השביעי כאשר תתחיל אחריו על הדרך הזה | ||
|- | |- | ||
| − | |||
| − | |||
| − | |||
| | | | ||
| − | {| | + | *Among the properties of these terms: the sum of two, which is the first even-times-odd number, with every term that is denominated by a square number, yields a square number. |
| + | :<math>\scriptstyle a_1+a_{n^2}=2+a_{n^2}=m^2</math> | ||
| + | |style="text-align:right;"|<big>ומסגלת</big> אלו המדרגו' שמקובץ השנים והוא הראשון שבזוג הנפרד עם כל מדרגה תהיה נקראת על שם מספר מרובע יוציא מספר מרבע | ||
|- | |- | ||
| − | | | + | | |
| + | ::Such as: | ||
| + | ::*<math>\scriptstyle{\color{blue}{2+a_4=2+14}}</math> | ||
| + | |style="text-align:right;"|כמו קבוצם עם הרביעי מהם והוא ארבעה עשר | ||
|- | |- | ||
| − | | | + | | |
| + | ::*<math>\scriptstyle{\color{blue}{2+a_9=2+34}}</math> | ||
| + | |style="text-align:right;"|ועם התשיעי מהם והוא ארבעה ושלשים | ||
|- | |- | ||
| − | | | + | | |
| + | :Also, the successive term after two, which is six, and it is the second even-times-odd number, when it is summed with every term, beginning from one, that is denominated by a square number, the sum is a square number. | ||
| + | :<math>\scriptstyle a_2+a_{n^2}=6+a_{n^2-1}=m^2</math> | ||
| + | |style="text-align:right;"|והנמשך אל שנים והוא הששה והוא זוג הנפרד השני כאשר יקובץ אל מספר כל {{#annot:term|1344,1578|6UFr}}מדרגה{{#annotend:6UFr}} מתחלת מן האחד שתהיה נקראת על שם מספר מרבע הנה יהיה המקובץ מרבע | ||
|- | |- | ||
| − | | | + | | |
| + | ::Such as: | ||
| + | ::*<math>\scriptstyle{\color{blue}{6+a_{4-1}=6+10}}</math> | ||
| + | |style="text-align:right;"|כמו הששה עם הרביעי והוא עשרה | ||
|- | |- | ||
| − | | | + | | |
| + | ::*<math>\scriptstyle{\color{blue}{6+a_{9-1}=6+30}}</math> | ||
| + | |style="text-align:right;"|ועם התשיעי והוא שלשים | ||
|- | |- | ||
| − | | | + | | |
| − | + | *The product of the name of every term by four, when the first number is subtracted from it, is the value of that term. | |
| − | + | :<math>\scriptstyle2\sdot\left(2n-1\right)=a_n=4n-2</math> | |
| + | |style="text-align:right;"|ומזה שהכאת הנקרא בשם כל מדרגה בארבעה יהיה כאשר תגרע ממנו המספר הראשון יהיה מספר אותה המדרגה | ||
|- | |- | ||
| | | | ||
| − | + | ::For instance: <math>\scriptstyle{\color{blue}{a_4=\left(4\sdot4\right)-2=16-2=14}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|המשל בזה שהבית הרביעי נקרא בשם ארבעה וכאשר נכפל בארבעה יהיה ששה עשר תפיל מהם המספר הראשון והוא שנים הנה יהיה ארבעה עשר |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :It can be reversed by saying that every term of them, when two is added to it, then divided by four, the result is the number of its term from the first. |
| + | :<math>\scriptstyle n=\frac{\left[2\sdot\left(2n-1\right)\right]+2}{4}=\frac{a_n+2}{4}</math> | ||
| + | |style="text-align:right;"|וכבר אפשר לך שתהפך זה ותאמר שכל מספר מהם כאשר תוסיף עליו שנים ותחלק על ארבעה הנה מה שיצא הוא מספר מדרגתו מן הראשון | ||
|- | |- | ||
| | | | ||
| − | *the | + | *Double the product of the number of the terms by itself is equal to the sum of the terms. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle2n^2=\sum_{i=1}^n \left[2\sdot\left(2i-1\right)\right]</math> |
| + | |style="text-align:right;"|ומזה שכפל הכאת מספר המדרגות בעצמם שוה למקובץ מספרם | ||
|- | |- | ||
| | | | ||
| − | : | + | ::For four terms: <math>\scriptstyle{\color{blue}{2\sdot4^2=32=2+6+10+14}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והנה תהיינה המדרגות ארבעה וכפל הכאתם בעצמם שנים ושלשים והנה זה מקובץ שנים ששה עשרה ארבעה עשר |
|- | |- | ||
| | | | ||
| − | * | + | *The sum of the first and the second is a cube, 8, [<math>\scriptstyle{\color{blue}{2+6=8}}</math>], and its term is already known, also the cube of the cube and so on [??] |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ומזה שמקובץ הראשון והשני מעקב ח' ואתה תדענו ותדע מדרגתו ממה שכבר ידעת ועוד מעקב מעקבו ועל הדרך הזה‫<ref>marg. לא הבנתי זאת הסגולה ואולי ירצה שקבוץ כל מספרי זוג הנפרד על הסדר שוה לכפלי המרובעי' הטבעיים על הסדר כי קבוץ מספרי ב' ו' שוה לכפל מרובע ב' וקבוץ ב' ו' י' שוה לכפל מרובע ג' וקבוץ ב' ו' י' י"ד שוה לכפל מרובע ד' וכן תמיד על זה הסדר א"ב ד"ל</ref> |
|- | |- | ||
| | | | ||
| − | * | + | *Constructing a square [table] of six by six from the even-times-odd numbers |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והנה נבנה מזוג הנפרד הנמשכים מרבע ששה בששה |
|- | |- | ||
| | | | ||
| − | + | :{|class="wikitable" style="color: blue; text-align:center;" | |
| − | + | |- | |
| − | + | |22||18||14||10||6||2 | |
| + | |- | ||
| + | |46||42||38||34||30||26 | ||
| + | |- | ||
| + | |70||66||62||58||54||50 | ||
| + | |- | ||
| + | |94||90||86||82||78||74 | ||
| + | |- | ||
| + | |118||114||110||106||102||98 | ||
| + | |- | ||
| + | |142||138||134||130||126||122 | ||
|} | |} | ||
| − | {| | + | |
| + | | | ||
| + | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
| + | |- | ||
| + | |כב||יח||יד||י||ו||ב | ||
| + | |- | ||
| + | |מו||מב||לח||לד||ל||כו | ||
| + | |- | ||
| + | |ע||סו||סב||נח||נד||נ | ||
| + | |- | ||
| + | |צד||צ||פו||פב||עח||עד | ||
| + | |- | ||
| + | |קיח||קיד||קי||קו||קב||צח | ||
|- | |- | ||
| − | | | + | |קמב||קלח||קלד||קל||קכו||קכב |
| − | + | |} | |
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | * | + | :*Among the properties of this square table: the units of the beginning of each row breadthwise are the same as the units of its end. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ומסגלות זה הלוח המרבע שאחדי ראשית התחלת כל שורה ברוחב כמו אחדי הסוף |
|- | |- | ||
| | | | ||
| − | + | ::If there is a zero in one of [the extremes], there is a zero in the other [extreme] as well. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואם יהיה באחד מהם גלגל הנקרא {{#annot:term|1554,205|ONwA}}ספרא{{#annotend:ONwA}} הנה באחר גלגל גם כן |
|- | |- | ||
| | | | ||
| − | * | + | :*the sum of the two extremes on the principal diagonal is equal to the sum of the two extremes on the other principal diagonal. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ומהם שמקובץ שני קצוות הקטר האחר שוה למקובץ שני קצות הקטר האחר |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :::Such as: <math>\scriptstyle{\color{blue}{2+142=22+122}}</math> |
| + | |style="text-align:right;"|כמו ב' עם קמ"ב שהם שני קצות הקטר וכ"ב עם קכ"ב והם קצות הקטר האחר | ||
|- | |- | ||
| | | | ||
| − | * | + | :*The sum of the extremes on the principal diagonal always has a root. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ומהם שמקובץ קצות הקטר נגדר לעולם |
|- | |- | ||
| | | | ||
| − | :<span style="color:#0000FF> | + | :*The sum of two numbers at the same distance from the two extremes on the principal diagonal is equal to the sum of the two extremes on the principal diagonal, and therefore it also has a root. |
| + | |style="text-align:right;"|ומהם שכל שני מספרים מרחקם משני קצות הקטר מרחק אחד הנה מקובצם שוה למקובץ שתי קצות הקטר והוא לזה נגדר גם כן | ||
| + | |- | ||
| + | | | ||
| + | :*The excess of each [consecutive] line over the beginning of the [preceding] line is the same. | ||
| + | |style="text-align:right;"|ומזה שתוספת כל שורה על התחלת זאת השורה אחד | ||
| + | |- | ||
| + | | | ||
| + | :::For the excess of [46 over 22] is as the excess of [70 over 46]: <math>\scriptstyle{\color{blue}{46-22=70-46}}</math> | ||
| + | |style="text-align:right;"|כי תוספת כ"ב על מ"ו כתוספת מ"ו על ע‫' | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ====== Even-Times-Even-Times Odd ====== | ||
| + | |||
| + | |style="text-align:right;"|<big>ואמנם</big> עניני {{#annot:term|1335,71|QJO8}}זוג הזוג והנפרד{{#annotend:QJO8}} הנה נדבר בהם | ||
| + | |- | ||
| + | | | ||
| + | *It is said to be similar to the even-times-odd, since it does not admit the repeated halving until reaching 1, except by a fraction [= not divisible by 2 repeatedly]. | ||
| + | |style="text-align:right;"|ונאמר שהוא דומה לזוג הנפרד באשר הוא איננו מקבל ההחצות ההולך בהדרגה בהתמדה עד האחד מזולת שבר | ||
| + | |- | ||
| + | | | ||
| + | *It is similar to the even-times-even, since its first halving does not yield two odds, and its halving does not cease by another division [= divisible by 2 more than once] | ||
| + | |style="text-align:right;"|וידמה לזוג הזוג באשר הוא לא יחצה החצותו הראשון אל שני נפרדים ולא יעמד החצותו אצל חלוקה אחרת | ||
| + | |- | ||
| + | | | ||
| + | *Its production is by multiplying the even-times-even numbers, beginning from four, by the successive odd numbers. | ||
| + | :<math>\scriptstyle2^m\sdot\left(2n-1\right)</math> | ||
| + | |style="text-align:right;"|ואמנם התילדותו הנה הוא מהכאת זוג הזוג המתחילים מן הארבע בנפרדים הנמשכים | ||
| + | |- | ||
| + | | | ||
| + | :The greater this even number is, the more it admits halving. | ||
| + | |style="text-align:right;"|וכל מה שהיה זה הזוג יותר רב יהיה קבלתו להחצות יותר | ||
| + | |- | ||
| + | | | ||
| + | *[Among the even-times-even-times-odd numbers] there are superabundant, deficient, and perfect numbers: | ||
| + | |style="text-align:right;"|וכבר יהיו בו הנוסף והחסר והשלם | ||
| + | |- | ||
| + | | | ||
| + | ::*<span style="color:#0000FF>68</span> is deficient number, and it is [an even-times-even-times-odd number] | ||
|style="text-align:right;"|כי השמנה וששים מספר חסר והוא מכללו | |style="text-align:right;"|כי השמנה וששים מספר חסר והוא מכללו | ||
|- | |- | ||
| | | | ||
| − | :<span style="color:#0000FF> | + | ::*The perfect number, such as: <span style="color:#0000FF>28</span>. |
|style="text-align:right;"|ואמנם השלם הנה כמו השמנה ועשרים | |style="text-align:right;"|ואמנם השלם הנה כמו השמנה ועשרים | ||
|- | |- | ||
| | | | ||
| − | :<span style="color:#0000FF> | + | ::*The superabundant number, such as: <span style="color:#0000FF>12</span> |
|style="text-align:right;"|והנוסף בו הרבה כמו השנים עשר | |style="text-align:right;"|והנוסף בו הרבה כמו השנים עשר | ||
|- | |- | ||
| | | | ||
| − | * | + | *[Among the even-times-even-times-odd numbers] there are also squares: |
|style="text-align:right;"|וכבר יפלו בו המרבעים ג"כ | |style="text-align:right;"|וכבר יפלו בו המרבעים ג"כ | ||
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle\left[2\sdot\left(2n-1\right)\right]^2</math> | + | :*The production of these squares that are [even-times-even-times-odd numbers] <math>\scriptstyle\left[2\sdot\left(2n-1\right)\right]^2</math>: |
|style="text-align:right;"|והתילדות אלו המרבעים אשר יפלו במספריהם | |style="text-align:right;"|והתילדות אלו המרבעים אשר יפלו במספריהם | ||
|- | |- | ||
| | | | ||
| − | : | + | ::*Multiplying the first even number by the first odd number, until they are six <math>\scriptstyle{\color{blue}{2\sdot3=6}}</math>, which is the root of the first square. |
|style="text-align:right;"|הוא שנכה הזוג הראשון בנפרד הראשון עד שיהיה ששה והנה הוא גדר המרבע הראשון | |style="text-align:right;"|הוא שנכה הזוג הראשון בנפרד הראשון עד שיהיה ששה והנה הוא גדר המרבע הראשון | ||
|- | |- | ||
| | | | ||
| − | : | + | ::*Multiplying it by the second odd number, until it is ten <math>\scriptstyle{\color{blue}{2\sdot5=10}}</math>, which is the root of the second square. |
| − | |style="text-align:right;"|אח"כ נכה אותו בנפרד השני עד שיהיה עשרה והנה הוא גדר המרבע השני ועל הדרך הזה | + | |style="text-align:right;"|אח"כ נכה אותו בנפרד השני עד שיהיה עשרה והנה הוא גדר המרבע השני |
| + | |- | ||
| + | | | ||
| + | ::And so on. | ||
| + | |style="text-align:right;"|ועל הדרך הזה | ||
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle\left[2^m\sdot\left[\left[2\sdot\left(n+1\right)\right]-1\right]\right]-\left[2^m\sdot\left(2n-1\right)\right]=2^{m+1}</math> | + | *When an [even-times-even-times-odd] term is subtracted from its successive term, the result is an even-times-even number. |
| + | :<math>\scriptstyle\left[2^m\sdot\left[\left[2\sdot\left(n+1\right)\right]-1\right]\right]-\left[2^m\sdot\left(2n-1\right)\right]=2^{m+1}</math> | ||
|style="text-align:right;"|וכאשר תגרע הבית מאשר ימשך אליו יצא לך זוג הזוג | |style="text-align:right;"|וכאשר תגרע הבית מאשר ימשך אליו יצא לך זוג הזוג | ||
|- | |- | ||
| | | | ||
| − | : | + | ::Such as: <math>\scriptstyle{\color{blue}{20-12}}</math> |
|style="text-align:right;"|כמו השנים עשר מהעשרים | |style="text-align:right;"|כמו השנים עשר מהעשרים | ||
|- | |- | ||
| | | | ||
| − | + | :::This is for what is produced from the products of four by odd numbers. | |
| + | :::<math>\scriptstyle\left[4\sdot\left[\left[2\sdot\left(n+1\right)\right]-1\right]\right]-\left[4\sdot\left(2n-1\right)\right]</math> | ||
|style="text-align:right;"|וזה במה שהתילדותו מהכאת הארבעה בנפרדים | |style="text-align:right;"|וזה במה שהתילדותו מהכאת הארבעה בנפרדים | ||
|- | |- | ||
| | | | ||
| − | : | + | ::And such as: <math>\scriptstyle{\color{blue}{40-24}}</math> |
|style="text-align:right;"|וכמו הארבעה ועשרים מן הארבעים | |style="text-align:right;"|וכמו הארבעה ועשרים מן הארבעים | ||
|- | |- | ||
| | | | ||
| − | + | :::This is for what is produced from the products of eight by odd numbers. | |
| + | :::<math>\scriptstyle\left[8\sdot\left[\left[2\sdot\left(n+1\right)\right]-1\right]\right]-\left[8\sdot\left(2n-1\right)\right]</math> | ||
|style="text-align:right;"|וזה במה שהתילדותו מהכאת השמנה בנפרדים | |style="text-align:right;"|וזה במה שהתילדותו מהכאת השמנה בנפרדים | ||
|- | |- | ||
| − | | | + | |This is what is said on the properties of the types of the even-times-even numbers that are affected by the properties of the odd number. |
| − | |style="text-align:right;"|והנה זה מה שנאמר אותו בסגלות מיני זוג הזוג | + | |style="text-align:right;"|והנה זה מה שנאמר אותו בסגלות מיני זוג הזוג ונעתק אל מיני סגלות הנפרד |
| − | |||
| − | |||
| − | |||
|} | |} | ||
{| | {| | ||
| Line 1,414: | Line 1,643: | ||
====== The Two - is it an even-times-even number or an even-times-odd number? ====== | ====== The Two - is it an even-times-even number or an even-times-odd number? ====== | ||
| − | + | | | |
| − | |style="text-align:right;"|וכבר נשאר עלינו הדבור בראשון שבמספרים והוא | + | |- |
| + | |The discussion on the first of the numbers, which is two, still remains: is it an even-times-even number, or an even-times-odd number? | ||
| + | |style="text-align:right;"|<big>וכבר</big> נשאר עלינו הדבור בראשון שבמספרים והוא השנים האם הוא זוג הזוג או זוג הנפרד | ||
|- | |- | ||
| | | | ||
| − | *even-times-odd number | + | *It is considered as an even-times-odd number from the aspect that its halving does not end in an even number. |
|style="text-align:right;"|וכבר יחשב מצד שהוא לא יכלה בו ההחצות אל זוג שהוא זוג הנפרד | |style="text-align:right;"|וכבר יחשב מצד שהוא לא יכלה בו ההחצות אל זוג שהוא זוג הנפרד | ||
|- | |- | ||
| | | | ||
| − | *even-times-even | + | *Some considered it as an even-times-even number as well as an even-times-odd number,and as the beginning of both [types]. |
|style="text-align:right;"|והעבירו קצתם שיהיה זוג הזוג וזוג הנפרד יחד ושיהיה התחלה לשניהם | |style="text-align:right;"|והעבירו קצתם שיהיה זוג הזוג וזוג הנפרד יחד ושיהיה התחלה לשניהם | ||
|- | |- | ||
| − | | | + | |[According to the author]: |
| + | *a true even is the number that is divided into the odd number by halving. | ||
|style="text-align:right;"|והנראה אלי שזוג באמת הוא המספר המתחלק אל הנפרד אצל ההחצות | |style="text-align:right;"|והנראה אלי שזוג באמת הוא המספר המתחלק אל הנפרד אצל ההחצות | ||
|- | |- | ||
| | | | ||
| − | *even-times-odd number | + | *The true even-times-odd number is the divided into the odd number by halving. |
|style="text-align:right;"|וזוג הנפרד באמת הוא המתחלק אל הנפרד אצל ההחצות | |style="text-align:right;"|וזוג הנפרד באמת הוא המתחלק אל הנפרד אצל ההחצות | ||
|- | |- | ||
| | | | ||
| − | *even-times-even number | + | *The even-times-even number is that whose half is an even number, and each half is halved by an even number, except the one. |
|style="text-align:right;"|והנה הזוג הזוג הוא אשר חציו זוג וכל חצי יחצה אותו זוג זולת האחד | |style="text-align:right;"|והנה הזוג הזוג הוא אשר חציו זוג וכל חצי יחצה אותו זוג זולת האחד | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|ולא ימלט מהחצות זוג | + | *The even number is necessarily halved. |
| + | |style="text-align:right;"|ולא ימלט מהחצות זוג | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *The even-times-odd number is that whose half is an odd number, and cannot be halved. |
| + | |style="text-align:right;"|וזוג הנפרד הוא אשר חציו נפרד ולא יחצה | ||
|- | |- | ||
| | | | ||
| + | *The odd is either a number, or one, from the aspect of its being indivisible into two parts | ||
| + | |style="text-align:right;"|והנפרד יהיה מספר או יהיה אחד מצד שלא יחלק בשני חלקים | ||
| + | |- | ||
| + | |? | ||
|style="text-align:right;"|והזוג לא יהיה ולא מספר | |style="text-align:right;"|והזוג לא יהיה ולא מספר | ||
|- | |- | ||
| Line 1,449: | Line 1,687: | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|ואם ירצה אחד שנשים השנים ראויים לשני השמות יחד הנה ראוי שנשים גדר זוג הזוג שהוא אשר לא יחצה אל מספר נפרד וכן הם השנים אמנם החלוקה לא תהיה על דרך היושר | + | *If one wants to define the two as matching the two names together: |
| + | |style="text-align:right;"|ואם ירצה אחד שנשים השנים ראויים לשני השמות יחד | ||
| + | |- | ||
| + | | | ||
| + | :*The definition of the even-times-even number should be stated as that which cannot be halved into an odd number. | ||
| + | |style="text-align:right;"|הנה ראוי שנשים גדר זוג הזוג שהוא אשר לא יחצה אל מספר נפרד | ||
| + | |- | ||
| + | | | ||
| + | :But, the two are so, hence the categorization is not by adequacy. | ||
| + | |style="text-align:right;"|וכן הם השנים אמנם החלוקה לא תהיה על דרך היושר | ||
| + | |- | ||
| + | | | ||
| + | *If one wants to extract the two from both names together: | ||
| + | |style="text-align:right;"|ואם רצה שנוציא השנים משני השמות יחד | ||
| + | |- | ||
| + | | | ||
| + | :*The definition of the even-times-even number should be stated as that which is halved into an even number. | ||
| + | |style="text-align:right;"|הנה ראוי שנשים גדר זוג הזוג שהוא הנחצה אל מספר זוג | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*The definition of the even-times-odd number should be stated as that which is halved into an odd number. |
| + | |style="text-align:right;"|וגדר זוג הנפרד שהוא אשר יחצה אל מספר נפרד | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :But, the two do not match one of the two names according to the adequacy of the categorization. |
| + | |style="text-align:right;"|ולא יהיו השנים ראויים אל אחד משני השמות עם יושר החלוקה | ||
|} | |} | ||
{| | {| | ||
| Line 1,462: | Line 1,719: | ||
===== Types of Odd Numbers ===== | ===== Types of Odd Numbers ===== | ||
| − | + | | | |
| − | |style="text-align:right;"| | + | |- |
| + | |Now the properties of the types of odd numbers will be discussed: | ||
| + | |style="text-align:right;"|<big>ונדבר</big> עתה בעניני מיני הנפרד | ||
|- | |- | ||
| − | | | + | |Some of the odd numbers are prime and some are composite. |
| − | |style="text-align:right;"|והנפרד ממנו | + | |style="text-align:right;"|והנפרד ממנו ראשון וממנו מורכב |
|- | |- | ||
| − | | | + | |The composite may be relatively prime. |
| − | |style="text-align:right;"|והמורכב כבר יהיה | + | |style="text-align:right;"|והמורכב כבר יהיה {{#annot:term|78,1520|fysN}} ראשון בהקש אל זולתו{{#annotend:fysN}} |
|- | |- | ||
| − | | | + | |All this is already known. |
|style="text-align:right;"|וכבר ידעת כל זה | |style="text-align:right;"|וכבר ידעת כל זה | ||
|- | |- | ||
| Line 1,478: | Line 1,737: | ||
| | | | ||
| + | |- | ||
| + | |When wishing to extract the terms of the composite numbers, one should return to the tables of the successive odd numbers: | ||
| + | |style="text-align:right;"|וכאשר תרצה שתוציא מדרגות המורכבים בעצמם הנה תשוב אל לוחות המספרים הנפרדים הנמשכים | ||
|- | |- | ||
| | | | ||
| − | * | + | *After three, every third number is a composite number <math>\scriptstyle3\sdot\left(2n-1\right)</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והנה תמצא כל שלשה אחר השלשה מורכב |
|- | |- | ||
| | | | ||
| − | + | *After five, every fifth number is a composite number <math>\scriptstyle5\sdot\left(2n-1\right)</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכל חמשה אחר החמשה מורכב |
|- | |- | ||
| | | | ||
| − | + | *And so on endlessly. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכן אל בלתי תכלית |
|- | |- | ||
| | | | ||
| − | : | + | :*Example for the first [case]: <span style="color:#0000FF>9; 15; 21</span> |
|style="text-align:right;"|דמיון הראשון הט' והט"ו והכ"א | |style="text-align:right;"|דמיון הראשון הט' והט"ו והכ"א | ||
|- | |- | ||
| | | | ||
| − | : | + | :*Example for the second [case]: <span style="color:#0000FF>15; 25; 3[5]</span> |
| − | |style="text-align:right;"|דמיון השני הט"ו והכ"ה והל | + | |style="text-align:right;"|דמיון השני הט"ו והכ"ה והל' |
| + | |- | ||
| + | | | ||
| + | :Likewise when starting from seven and nine according to this way. | ||
| + | |style="text-align:right;"|וכמו כן כאשר תתחיל מן השבעה והתשעה על הדרך הזה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *One finds that three counts: |
| + | |style="text-align:right;"|ותמצא שם דבר והוא שהשלשה מהם ימנה | ||
|- | |- | ||
| | | | ||
| − | : | + | :*The first composite of their terms by the first number of the odds, as nine <math>\scriptstyle{\color{blue}{9=3\sdot3}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|המורכב הראשון אשר במדרגותיהם במספר ראשון הנפרדים והוא בעצמו כמו התשעה |
|- | |- | ||
| | | | ||
| − | : | + | :*The second by the consecutive odd number, which is five <math>\scriptstyle{\color{blue}{3\sdot5}}</math> |
|style="text-align:right;"|והשני בנפרד אשר ימשך אליו כמו החמשה | |style="text-align:right;"|והשני בנפרד אשר ימשך אליו כמו החמשה | ||
|- | |- | ||
| | | | ||
| − | : | + | :*The third by the third odd number, which is seven <math>\scriptstyle{\color{blue}{3\sdot7}}</math> |
|style="text-align:right;"|והשלישי בנפרד השלישי כמו השבעה‫<ref>marg. ר"ל ג' ימנה ט' בג' וימנה ט"ו בה' וימנה כ"א בז‫'</ref> | |style="text-align:right;"|והשלישי בנפרד השלישי כמו השבעה‫<ref>marg. ר"ל ג' ימנה ט' בג' וימנה ט"ו בה' וימנה כ"א בז‫'</ref> | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{15=5\sdot3}}</math> | + | *Five also counts: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והחמשה ג"כ ימנה |
| + | |- | ||
| + | | | ||
| + | :*The its consecutive by the first number of the odds, which is three, as fifteen <math>\scriptstyle{\color{blue}{15=5\sdot3}}</math> | ||
| + | |style="text-align:right;"|אשר ימשך אליו בראשון בנפרדים והוא השלשה כמו החמשה עשר | ||
|- | |- | ||
| | | | ||
| − | : | + | :*The second by itself, as twenty five <math>\scriptstyle{\color{blue}{25=5\sdot5}}</math> |
|style="text-align:right;"|והשני בעצמו כמו החמשה ועשרים | |style="text-align:right;"|והשני בעצמו כמו החמשה ועשרים | ||
|- | |- | ||
| | | | ||
| − | : | + | :*The third by its consecutive [odd], which is seven, as thirty five <math>\scriptstyle{\color{blue}{35=5\sdot7}}</math> |
| − | |style="text-align:right;"|והשלישי | + | |style="text-align:right;"|והשלישי במה שאחריו כמו החמשה ושלשים כי הוא ימנה אותם בשבעה‫<ref>marg. הה' ימנה ט"ו בג' וכ"ה בה' ול"ה בז‫'</ref> |
|} | |} | ||
{| | {| | ||
| Line 1,531: | Line 1,802: | ||
====== Relatively Prime Numbers ====== | ====== Relatively Prime Numbers ====== | ||
| − | + | | | |
| − | | | ||
|- | |- | ||
| | | | ||
| − | + | :As for the composite in relation to itself that is prime in relation to the other: | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואמנם המורכב בעצמו ו{{#annot:term|78,1520|a04n}}ראשון אצל זולתו{{#annotend:a04n}} |
|- | |- | ||
| | | | ||
| + | :It is every square of a prime number in relation to another square of a prime number among these successive odd numbers. | ||
| + | :[= if p and q are prime numbers, then <math>\scriptstyle p^2</math> and <math>\scriptstyle q^2</math> are relatively prime] | ||
| + | |style="text-align:right;"|הנה הוא כל מרבע {{#annot:term|78,1520|Ie1G}}ראשון בהקש אל{{#annotend:Ie1G}} מרבע ראשון אחר מאלו הנפרדים הנמשכים‫<ref>marg. א"ב ר"ל כל מרבע מספר ראשון בהקש אל מרבע מספר ראשון</ref> | ||
| + | |- | ||
| + | |This is what is said about the properties of the even and the odd numbers. | ||
|style="text-align:right;"|והנה זה מה שנאמר אותו בעניני הזוג והנפרד | |style="text-align:right;"|והנה זה מה שנאמר אותו בעניני הזוג והנפרד | ||
|- | |- | ||
| Line 1,544: | Line 1,819: | ||
==== Superabundant / Deficient / Perfect Numbers ==== | ==== Superabundant / Deficient / Perfect Numbers ==== | ||
| − | + | | | |
| − | |style="text-align:right;"|והמספר חלוקה אחרת ממנו נוסף וממנו חסר וממנו שלם | + | |- |
| + | |Another categorization of the number: some number are superabundant, some are deficient, and some are perfect. | ||
| + | |style="text-align:right;"|<big>והמספר</big> חלוקה אחרת ממנו נוסף וממנו חסר וממנו שלם | ||
| + | |- | ||
| + | |All this is already known, as well as the quality of the production of the perfect number from the even-times-even numbers. | ||
| + | |style="text-align:right;"|וכבר ידעת כל זה וידעת איכות התילד המספר השלם מזוגי הזוגות‫<ref>marg. מסוף ט' לאקלידס</ref> | ||
| + | |- | ||
| + | |The perfect number is an even number only, because it is produced from the multiplication of an odd number by an even number. | ||
| + | |style="text-align:right;"|ודע שהמספר השלם לא יהיה אלא זוג לפי שהוא אמנם נתילד מהכאת נפרד בזוג | ||
|- | |- | ||
| | | | ||
| − | == | + | :*There is one [perfect number] in the units: <span style="color:#0000FF>6</span> |
| − | + | |style="text-align:right;"|ונזדמן שהנופל ממנו באחדים אחד והוא ששה | |
| + | |- | ||
| | | | ||
| + | :*One in the tens: <span style="color:#0000FF>28</span> | ||
| + | |style="text-align:right;"|ובעשרות אחד והוא שמנה ועשרים | ||
|- | |- | ||
| | | | ||
| − | * | + | :*One in the hundreds: <span style="color:#0000FF>496</span> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ובמאות אחד והוא תצ"ו |
|- | |- | ||
| | | | ||
| − | * | + | :*One in the thousands: <span style="color:#0000FF>8128</span> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ובאלפים אחד והוא שמנה אלפים וקכ"ח |
|- | |- | ||
| | | | ||
| − | *in each rank there is one perfect number | + | *And so on, in each rank there is one [perfect number] |
| − | + | |style="text-align:right;"|וכמו כן בכל מין אחד | |
| − | |||
| − | |||
| − | |||
| − | |style="text-align:right;"| | ||
| − | |||
| − | |||
| − | |||
| − | וכמו כן בכל מין אחד | ||
|- | |- | ||
| | | | ||
| − | * | + | *The units [of a perfect number] are necessarily 6 or 8, even if it does not determined by the experience that they are recurring repeatedly. |
|style="text-align:right;"|לא ימלט מאחדים הם ששה ושמנה ואם לא יתחיב אצל הנסיון בהם שיהיו חוזרים חלילה | |style="text-align:right;"|לא ימלט מאחדים הם ששה ושמנה ואם לא יתחיב אצל הנסיון בהם שיהיו חוזרים חלילה | ||
|- | |- | ||
| | | | ||
| − | *the | + | *Among the properties of the perfect number: when it is multiplied by eight and one is added to [the product], [the result] has a root. |
| + | :[If a is a perfect number → 8a+1 is a square number] | ||
|style="text-align:right;"|ומסגלות המספר השלם שכאשר הוכה בשמנה ונוסף עליו אחד יהיה נגדר | |style="text-align:right;"|ומסגלות המספר השלם שכאשר הוכה בשמנה ונוסף עליו אחד יהיה נגדר | ||
|- | |- | ||
| | | | ||
| − | + | :When its root is divided by four and a quarter is added to the quotient, it is an even-times-even number, such that when multiplied by its double minus one the product is the perfect number. | |
| + | :[If a is a perfect number → <math>\scriptstyle\frac{\sqrt{8a+1}}{4}+\frac{1}{4}</math> is an even-times-even number; and <math>\scriptstyle\left(\frac{\sqrt{8a+1}}{4}+\frac{1}{4}\right)\sdot\left[\left[2\sdot\left(\frac{\sqrt{8a+1}}{4}+\frac{1}{4}\right)\right]-1\right]=a</math>] | ||
|style="text-align:right;"|וכאשר יחלק גדרו על ארבעה ויתוסף על מה שיתקבץ רביע יהיה זוג הזוג אשר הוכה בכפלו זולת אחד עד שיצא זה המספר השלם | |style="text-align:right;"|וכאשר יחלק גדרו על ארבעה ויתוסף על מה שיתקבץ רביע יהיה זוג הזוג אשר הוכה בכפלו זולת אחד עד שיצא זה המספר השלם | ||
|- | |- | ||
| | | | ||
| − | :Example:<math>{\color{blue} | + | ::Example: <math>\scriptstyle{\color{blue}{\left(8\sdot6\right)+1=49}}</math> |
| − | |style="text-align:right;"|המשל בזה ששה בשמנה נוסף עליו אחד הוא מ"ט | + | |style="text-align:right;"|המשל בזה ששה בשמנה נוסף עליו אחד הוא מ"ט |
|- | |- | ||
| | | | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{\sqrt{49}}{4}+\frac{1}{4}=\frac{7}{4}+\frac{1}{4}\left(1+\frac{3}{4}\right)+\frac{1}{4}=2}}</math> is an even-times-even number | ||
| + | |style="text-align:right;"|וגדרו שבעה ורביעיתו אחד ושלשה רביעיו' וכאשר יתוסף עליו רביע יהיה שנים והוא זוג הזוג | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{2\sdot\left[\left(2\sdot2\right)-1\right]=6}}</math> | ||
| + | |style="text-align:right;"|אשר נפלה ההכאה בכפלו זולת אחד כאשר יצא ששה‫<ref>marg. א"ב ר"ל כי יציאת ששה היתה כאשר לקחנו שנים זוג הזוג והאחד ונתקבץ שלושה מספר ראשון והכינו אותו בשנים ויצא ששה מסוף ט' לאקליד‫'</ref> | ||
| + | |- | ||
| + | |The superabundant and the deficient numbers are as explained in every chapter [?] | ||
|style="text-align:right;"|אמנם המספר הנוסף והחסר הנה יהיו כמו שכבר נתבאר בכל שער | |style="text-align:right;"|אמנם המספר הנוסף והחסר הנה יהיו כמו שכבר נתבאר בכל שער | ||
|- | |- | ||
| | | | ||
| + | *Some people applied an examination for the extraction of the perfect, superabundant and the deficient numbers: | ||
|style="text-align:right;"|והנה בהוצאת השלם והחסר והנוסף בחינה נפלה לקצת האנשים | |style="text-align:right;"|והנה בהוצאת השלם והחסר והנוסף בחינה נפלה לקצת האנשים | ||
|- | |- | ||
| | | | ||
| − | + | :For every even-times-even number, when it is multiplied by a prime number, whichever it may be: | |
| − | |style="text-align:right;"|והיא שכל זוג הזוג כאשר הוכה במספר ראשון איך שיהיה | + | |style="text-align:right;"|והיא שכל זוג הזוג כאשר הוכה במספר ראשון איך שיהיה |
|- | |- | ||
| | | | ||
| − | : | + | :*If the even-times-even number is greater than half this prime number by one half, the product is always a perfect number. |
| − | + | ::[If <math>\scriptstyle2^n-\left(\frac{1}{2}p\right)=\frac{1}{2}</math> → <math>\scriptstyle2^n\sdot p</math> is a perfect number] | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אחר שיהיה זוג הזוג יותר מחצי זה הראשון בחצי אחד הנה המתקבץ ממנו לעולם מספר שלם |
| − | |||
|- | |- | ||
| | | | ||
| − | *<math>\ | + | :::Such as: |
| − | |style="text-align:right;"| | + | :::*<math>\scriptstyle{\color{blue}{2\sdot3}}</math> |
| + | |style="text-align:right;"|כמו השנים בשלשה | ||
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle2^n-\left(\frac{1}{2}p\right) | + | :::*<math>\scriptstyle{\color{blue}{4\sdot7}}</math> |
| + | |style="text-align:right;"|והארבעה בשבעה | ||
| + | |- | ||
| + | | | ||
| + | :*If it is greater than its half by more than one half, the product is always a superabundant number. | ||
| + | ::[If <math>\scriptstyle2^n-\left(\frac{1}{2}p\right)>\frac{1}{2}</math> → <math>\scriptstyle2^n\sdot p</math> is a superabundant number] | ||
| + | |style="text-align:right;"|ואם יהיה יותר מחציו ביותר מחצי אחד הנה המתקבץ ממנו לעולם מספר נוסף | ||
| + | |- | ||
| + | | | ||
| + | :*If less than its half, whichever the deficiency is, [the product is always] a deficient number. | ||
| + | ::[If <math>\scriptstyle2^n<\frac{1}{2}p</math> → <math>\scriptstyle2^n\sdot p</math> is a deficient number] | ||
|style="text-align:right;"|ואם יהיה פחות מחציו איך שיהיה הפחת הנה המספר חסר | |style="text-align:right;"|ואם יהיה פחות מחציו איך שיהיה הפחת הנה המספר חסר | ||
|- | |- | ||
| | | | ||
| − | : | + | ::*The example for the first [case]: <math>\scriptstyle{\color{blue}{4\sdot5}}</math> [<span style="color:#0000FF>superabundant</span>] |
|style="text-align:right;"|משל הראשון הנה ארבעה בחמשה | |style="text-align:right;"|משל הראשון הנה ארבעה בחמשה | ||
|- | |- | ||
| | | | ||
| − | : | + | ::*The example for the second [case]: <math>\scriptstyle{\color{blue}{4\sdot11}}</math> [<span style="color:#0000FF>deficient</span>] |
|style="text-align:right;"|ומשל השני הארבעה באחד עשר | |style="text-align:right;"|ומשל השני הארבעה באחד עשר | ||
|- | |- | ||
| | | | ||
| + | *For every number of the perfect numbers that is multiplied by a prime number, such that this prime number is not a divisor of the perfect number: | ||
|style="text-align:right;"|וכל מספר מהמספרים השלמים הוכה במספר ראשון ולא ימנה זה המספר הראשון זה המספר השלם | |style="text-align:right;"|וכל מספר מהמספרים השלמים הוכה במספר ראשון ולא ימנה זה המספר הראשון זה המספר השלם | ||
|- | |- | ||
| | | | ||
| − | : | + | ::Such as: <math>\scriptstyle{\color{blue}{6\sdot7=42}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כמו הששה כאשר הוכה בשבעה ונתחדש מ"ב |
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{\frac{1}{2}\sdot42=21}}</math> | ||
| + | |style="text-align:right;"|ולו מן החלקים החצי והוא כ"א | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{\frac{1}{3}\sdot42=14}}</math> | ||
| + | |style="text-align:right;"|ושליש והוא י"ד | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{\frac{1}{6}\sdot42=7}}</math> | ||
| + | |style="text-align:right;"|והששית והוא ז' | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{\frac{1}{7}\sdot42=6}}</math> | ||
| + | |style="text-align:right;"|והשביעית והוא ו' | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{\frac{1}{14}\sdot42=3}}</math> | ||
| + | |style="text-align:right;"|וחלק מי"ד והוא ג' | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{\frac{1}{21}\sdot42=2}}</math> | ||
| + | |style="text-align:right;"|וחלק מכ"א והוא ב' | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{\frac{1}{42}\sdot42=1}}</math> | ||
| + | |style="text-align:right;"|וחלק ממ"ב והוא א' | ||
| + | |- | ||
| + | | | ||
| + | ::The total sum: <math>\scriptstyle{\color{blue}{21+14+7+6+3+2+1=54}}</math> | ||
| + | |style="text-align:right;"|וכלל זה נ"ד | ||
|- | |- | ||
| | | | ||
| − | * | + | ::It exceeds the 43 by 12, which is double the 6 <math>\scriptstyle{\color{blue}{54-42=12=2\sdot6}}</math> |
| + | |style="text-align:right;"|הנה מוסיף על מ"ב בי"ב והוא כפל ששה | ||
| + | |- | ||
| + | | | ||
| + | *Every number divisible by 2 is always deficient. | ||
|style="text-align:right;"|וכל מספר ימנהו שנים הנה הוא חסר לעולם | |style="text-align:right;"|וכל מספר ימנהו שנים הנה הוא חסר לעולם | ||
|- | |- | ||
| | | | ||
| − | * | + | *All the prime numbers are undoubtedly deficient. |
|style="text-align:right;"|וכל המספרים הראשוני' חסרי' בלי ספק | |style="text-align:right;"|וכל המספרים הראשוני' חסרי' בלי ספק | ||
|- | |- | ||
| | | | ||
| − | * | + | *All the even-times-even numbers are deficient by 1. |
|style="text-align:right;"|וכל זוגי הזוג חסרים באחד | |style="text-align:right;"|וכל זוגי הזוג חסרים באחד | ||
|- | |- | ||
| | | | ||
| − | * | + | *Every number divisible by 2 and 3 is always superabundant, except for the number 6. |
|style="text-align:right;"|וכל מספר זולת הששה ימנוהו השנים והשלשה הנה הוא נוסף לעולם | |style="text-align:right;"|וכל מספר זולת הששה ימנוהו השנים והשלשה הנה הוא נוסף לעולם | ||
|- | |- | ||
| | | | ||
| − | *every number divisible by two and two other numbers whose sum is divisible by three is superabundant [ | + | *every number divisible by two and two other numbers [whose sum is divisible by three] is superabundant [<span style="color:red>the wording is very vague</span>] |
|style="text-align:right;"|וכל מספר ימנה אותו השנים ושני מספרים יהיה קריאת מקובצם עומדת מקום השליש רצוני שיהיה אחריתם כמו השליש רצוני שיהיה החבור משני יחסי חלקיהם בנכח המוסיף שליש כמו מקובץ שני יחסי המוסיף חומש והמוסיף שביעית אשר הוא לנכח המוסיף שלישית הנה הוא נוסף לעולם | |style="text-align:right;"|וכל מספר ימנה אותו השנים ושני מספרים יהיה קריאת מקובצם עומדת מקום השליש רצוני שיהיה אחריתם כמו השליש רצוני שיהיה החבור משני יחסי חלקיהם בנכח המוסיף שליש כמו מקובץ שני יחסי המוסיף חומש והמוסיף שביעית אשר הוא לנכח המוסיף שלישית הנה הוא נוסף לעולם | ||
|- | |- | ||
| Line 1,693: | Line 2,029: | ||
|style="text-align:right;"|כמו מה שראשיתו שלשה אח"כ חמשה אח"כ שבעה אח"כ תשעה ומורכבם הוא תשע מאות וארבעים וחמשה והוא המספר הראשון שבנפרדים נוסף בשלישית ואם יניח זה ההמשך לא יתחיב שיהיה נוסף | |style="text-align:right;"|כמו מה שראשיתו שלשה אח"כ חמשה אח"כ שבעה אח"כ תשעה ומורכבם הוא תשע מאות וארבעים וחמשה והוא המספר הראשון שבנפרדים נוסף בשלישית ואם יניח זה ההמשך לא יתחיב שיהיה נוסף | ||
|- | |- | ||
| − | | | + | |The end of the discussion on this manner of arithmetic - moving on to the manner by which the relation of a number to a number is examined. |
|style="text-align:right;"|ונחתום הנה הדבור בזה האופן מחכמת המספר ונעתק אל האופן אשר יבחן ממנו בצרוף מספר אל מספר | |style="text-align:right;"|ונחתום הנה הדבור בזה האופן מחכמת המספר ונעתק אל האופן אשר יבחן ממנו בצרוף מספר אל מספר | ||
| − | |||
| − | |||
| − | |||
|} | |} | ||
{| | {| | ||
| Line 1,712: | Line 2,045: | ||
| | | | ||
|- | |- | ||
| − | | | + | |The number is examined by examination from the aspect of its attention to itself and to the properties that are necessary for it, to what is a number and what is a type of number. |
| − | |style="text-align:right;"|כבר יעויין במספר עיון מצד ההשגחה בעצמו ובעניינים | + | |style="text-align:right;"|כבר יעויין במספר עיון מצד ההשגחה בעצמו ובעניינים המתחייבים לו במה שהוא מספר ומה שהוא מין מספר |
|- | |- | ||
| − | | | + | |It is examined from other aspects, among them from the aspect of being related to another number. |
| − | |style="text-align:right;"|וכבר יעויין בו מצדדים | + | |style="text-align:right;"|וכבר יעויין בו מצדדים אחרים מהם מצד היותו מצטרף אל מספר אחר |
|- | |- | ||
| − | | | + | |This other number is either other from it by number and not by species, or part of the species, so the relation is a relation of changing. |
| − | |style="text-align:right;"|וזה המספר האחר | + | |style="text-align:right;"|וזה המספר האחר אם יהיה אחר ממנו במספר לא במין או חלק המין יהיה הצרוף צרוף השנוי |
|- | |- | ||
| − | | | + | |Every two changing terms - one of them is greater and the other is less |
|style="text-align:right;"|וכל שני משתנים הנה אחד מהם מוסיף והאחר חסר | |style="text-align:right;"|וכל שני משתנים הנה אחד מהם מוסיף והאחר חסר | ||
|- | |- | ||
| − | | | + | |When one knows the properties of the greater to the less, he knows the properties of the less to the greater, according to what the evenness requires in relation. |
|style="text-align:right;"|וכאשר תדע עניני המוסיף אצל החסר תדע עניני החסר אצל המוסיף כפי מה שיחיבהו היושר בהצטרפות | |style="text-align:right;"|וכאשר תדע עניני המוסיף אצל החסר תדע עניני החסר אצל המוסיף כפי מה שיחיבהו היושר בהצטרפות | ||
| + | |- | ||
| + | |The greater is either simple or not simple. | ||
| + | |style="text-align:right;"|והמוסיף אם פשוט ואם בלתי פשוט | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *The Simple is either double or multiple; either superparticular or superpartient |
| + | |style="text-align:right;"|והפשוט אם כפל ואם כפלים ואם מוסיף בחלק או חלקים | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | *The Compounded is the double superparticular, or the double superpartient, or the multiple superparticular, or the multiple superpartient. |
| + | |style="text-align:right;"|והמורכב הוא המוסיף בכפל והחלק או המוסיף בכפל והחלקים או המוסיף בכפלים והחלק או המוסיף בכפלים והחלקים | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | :[The multiple] and the superpartient meaning what is more than one duplication, or more than one part, even if it is [only] double, or two parts. |
| + | |style="text-align:right;"|והחלקים כונתנו בהם מה שהוא יותר מכפל אחד או חלק אחד ואם היה שני כפלים או שני חלקים | ||
|- | |- | ||
| − | | | + | |As for the less, the custom has spread to indicate it by sub-, as saying subsuperparticular |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והחסר הנה כבר פשט המנהג שיורו עליו באשר הוא אשר תחת ככה כאמרם אשר תחת המוסיף חלק |
|- | |- | ||
| − | | | + | |Sometimes its name is derived from the name of the number of the multiples, such as the third, the quarter, and the twelfth; and sometimes it is called by two ratios, as saying half the sixth, or fifth of a tenth. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ולפעמים יהיה נגזר לו שם משם מספר הכפלים כמו השליש והרביע והחלק משנים עשר ולפעמים יקרא בשני יחסים כאמרם חצי הששית חומש עשירית |
|- | |- | ||
| | | | ||
| Line 1,753: | Line 2,092: | ||
|- | |- | ||
| | | | ||
| − | + | *The first multiple is the double, in which the addition is one time the other. | |
| − | |style="text-align:right;"|והנה | + | |style="text-align:right;"|והנה הנכפל הראשון הוא הנכפל השניי והוא אשר התוספת בו בדמיון האחר |
|- | |- | ||
| | | | ||
| − | : | + | :*Its beginning is from the numbers one and two <math>\scriptstyle{\color{blue}{2:1}}</math> |
|style="text-align:right;"|והתחלתו במספרים מן האחד והשנים | |style="text-align:right;"|והתחלתו במספרים מן האחד והשנים | ||
|- | |- | ||
| | | | ||
| − | : | + | :The smaller are added by the sequence of the successive numbers |
|style="text-align:right;"|ויתוסף החסר על סדר המספרים הנמשכים | |style="text-align:right;"|ויתוסף החסר על סדר המספרים הנמשכים | ||
|- | |- | ||
| | | | ||
| − | : | + | :The greater, which are the doubles, by the sequence of the successive even numbers, exceeding two by two |
|style="text-align:right;"|והמוסיף והוא הכפל על סדר הזוגות הנמשכים בהעדף שנים שנים | |style="text-align:right;"|והמוסיף והוא הכפל על סדר הזוגות הנמשכים בהעדף שנים שנים | ||
|- | |- | ||
| | | | ||
| − | + | *Then the triple, in which the addition is two times. | |
| − | |style="text-align:right;"|אח"כ | + | |style="text-align:right;"|אח"כ הנכפל השלישיי והוא אשר התוספת בו בשני דמיונים |
|- | |- | ||
| | | | ||
| − | : | + | :*Its beginning is from three and one <math>\scriptstyle{\color{blue}{3:1}}</math> |
|style="text-align:right;"|והתחלתו מהשלשה והאחד | |style="text-align:right;"|והתחלתו מהשלשה והאחד | ||
|- | |- | ||
| | | | ||
| − | : | + | :The smaller are added by the sequence of the successive numbers |
|style="text-align:right;"|ויתוסף החסר על סדר המספרים הנמשכים | |style="text-align:right;"|ויתוסף החסר על סדר המספרים הנמשכים | ||
|- | |- | ||
| | | | ||
| − | : | + | :The greater [are added] three by three |
| − | |style="text-align:right;"|והנוסף בשלשה שלשה | + | |style="text-align:right;"|והנוסף בשלשה שלשה |
|- | |- | ||
| | | | ||
| − | + | ::Such as: <math>\scriptstyle{\color{blue}{3;\ 6;\ 9;\ 12}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כמו שלשה וששה ותשעה ושנים עשר |
|- | |- | ||
| − | | | + | |According to this way the less are added one by one in all the multiple ratios and the greater by the number of the multiples. |
| − | + | |style="text-align:right;"|ועל הדרך הזה יתוסף החסר בכל היחסים בעלי הכפל באחד אחד והנוסף במספר הכפלים | |
| − | |style="text-align:right;"|והנוסף במספר הכפלים | ||
|- | |- | ||
| − | | | + | |The beginning of the less is from 1. |
| − | |||
|style="text-align:right;"|ותהיה התחלת החסר מן האחד | |style="text-align:right;"|ותהיה התחלת החסר מן האחד | ||
|- | |- | ||
| − | | | + | |The beginning of the greater from the number by which the number of the multiple is denominated [= the multiplier]. |
| − | |||
|style="text-align:right;"|והתחלת הנוסף מהמספר אשר על שמו יקרא מספר הכפלים | |style="text-align:right;"|והתחלת הנוסף מהמספר אשר על שמו יקרא מספר הכפלים | ||
| + | |} | ||
| + | {| | ||
|- | |- | ||
| | | | ||
| + | |||
===== Superparticular Ratio ===== | ===== Superparticular Ratio ===== | ||
| Line 1,806: | Line 2,145: | ||
|- | |- | ||
| | | | ||
| − | + | *The first of the superparticular is that which adds to the other its half [= sesquialter]. | |
| − | |style="text-align:right;"|והראשון אשר ב | + | |style="text-align:right;"|והראשון אשר ב{{#annot:term|1659,125|6mUG}}מוסיף חלק{{#annotend:6mUG}} הוא המוסיף על האחר בכמו חציו |
|- | |- | ||
| | | | ||
| − | : | + | :*Its beginning is from three and two <math>\scriptstyle{\color{blue}{3:2}}</math> |
|style="text-align:right;"|והתחלתו מהשלשה והשנים | |style="text-align:right;"|והתחלתו מהשלשה והשנים | ||
|- | |- | ||
| | | | ||
| − | : | + | :The less are added by the sequence of the successive even numbers, since they have a half [= divisible by 2]. |
|style="text-align:right;"|ויתוסף החסר על סדר הזוגות הנמשכים לפי שיש להם חצי | |style="text-align:right;"|ויתוסף החסר על סדר הזוגות הנמשכים לפי שיש להם חצי | ||
|- | |- | ||
| | | | ||
| − | : | + | :The greater [are added] three by three. |
|style="text-align:right;"|והמוסיף בשלשה שלשה | |style="text-align:right;"|והמוסיף בשלשה שלשה | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::Such as: <math>\scriptstyle{\color{blue}{3:2;\quad6:4;\quad9:6}}</math> |
|style="text-align:right;"|כמו השנים עם השלשה אח"כ הארבעה עם הששה אח"כ הששה עם התשעה | |style="text-align:right;"|כמו השנים עם השלשה אח"כ הארבעה עם הששה אח"כ הששה עם התשעה | ||
|- | |- | ||
| | | | ||
| − | + | *After the sesquialter is the sesquitertian | |
| − | |style="text-align:right;"|ואחר המוסיף חצי הוא המוסיף שליש | + | |style="text-align:right;"|ואחר {{#annot:sesquialter|125|29QX}}המוסיף חצי{{#annotend:29QX}} הוא {{#annot:sesquitertian|125|OyXe}}המוסיף שליש{{#annotend:OyXe}} |
|- | |- | ||
| | | | ||
| − | : | + | :*Its beginning is from four and three <math>\scriptstyle{\color{blue}{4:3}}</math> |
|style="text-align:right;"|והתחלתו מן הארבעה והשלשה | |style="text-align:right;"|והתחלתו מן הארבעה והשלשה | ||
|- | |- | ||
| | | | ||
| − | : | + | :The less are added three by three. |
| − | |style="text-align:right;"|ויתוסף החסר בשלשה שלשה | + | |style="text-align:right;"|ויתוסף החסר בשלשה שלשה |
|- | |- | ||
| | | | ||
| − | ::greater | + | ::Such as: <math>\scriptstyle{\color{blue}{3;\ 6;\ 9}}</math> |
| + | |style="text-align:right;"|כמו השלשה והששה והתשעה | ||
| + | |- | ||
| + | | | ||
| + | :The greater [are added] four by four. | ||
|style="text-align:right;"|והמוסיף בארבעה ארבעה | |style="text-align:right;"|והמוסיף בארבעה ארבעה | ||
|- | |- | ||
| − | | | + | |According to this way the matter proceeds always by this order. |
|style="text-align:right;"|ועל הדרך הזה ילך הענין תמיד על זה הסדר | |style="text-align:right;"|ועל הדרך הזה ילך הענין תמיד על זה הסדר | ||
| + | |} | ||
| + | {| | ||
|- | |- | ||
| | | | ||
| + | |||
===== Table of Multiple and Superparticular Ratios ===== | ===== Table of Multiple and Superparticular Ratios ===== | ||
| | | | ||
|- | |- | ||
| − | | | + | |Setting a square table with lines, starting from one, the beginnings of its lines are added lengthwise and breadthwise by the sequence of the natural numbers |
| − | |style="text-align:right;"|והנה כאשר נרשום לוח בעל טורים מרובע יתחיל מן האחד ויתוספו ראשי שורותיו בארך וברחב על סדר המספרים הטבעיים ועל הדרך הזה תניח | + | |style="text-align:right;"|והנה כאשר נרשום לוח בעל טורים מרובע יתחיל מן האחד ויתוספו ראשי שורותיו בארך וברחב על סדר המספרים הטבעיים |
| + | |- | ||
| + | |By this way these ratios are established in it, as well as other propositions that are derived from them. | ||
| + | |style="text-align:right;"|ועל הדרך הזה תניח בו אלה היחסים ומשפטים אחרים יוצאים מהם | ||
|- | |- | ||
| | | | ||
| + | :Let there be a table of ten by ten lines: | ||
|style="text-align:right;"|והנה יהיה זה הלוח הבעל טורים עשרה על עשרה | |style="text-align:right;"|והנה יהיה זה הלוח הבעל טורים עשרה על עשרה | ||
|- | |- | ||
| Line 1,913: | Line 2,263: | ||
|style="text-align:right;"|והשלישית על יחס השלשה כפלים | |style="text-align:right;"|והשלישית על יחס השלשה כפלים | ||
|- | |- | ||
| − | | | + | |By this way the excess is found as is said. |
|style="text-align:right;"|ועל הדרך הזה נמצא ההעדף כפי מה שנאמר לך | |style="text-align:right;"|ועל הדרך הזה נמצא ההעדף כפי מה שנאמר לך | ||
|- | |- | ||
| | | | ||
*[<math>\scriptstyle{\color{blue}{R_3:R_2}}</math>]: The third line is in the sesquialter ratio to the second line. | *[<math>\scriptstyle{\color{blue}{R_3:R_2}}</math>]: The third line is in the sesquialter ratio to the second line. | ||
| − | |style="text-align:right;"|ונמצא השורה השלישית אל השנית על יחס המוסיף חצי | + | |style="text-align:right;"|ונמצא השורה השלישית אל השנית על {{#annot:sesquialter|125|y0yz}}יחס המוסיף חצי{{#annotend:y0yz}} |
|- | |- | ||
| | | | ||
*[<math>\scriptstyle{\color{blue}{R_4:R_3}}</math>]: The fourth [line] is in the sesquitertian ratio to the third [line]. | *[<math>\scriptstyle{\color{blue}{R_4:R_3}}</math>]: The fourth [line] is in the sesquitertian ratio to the third [line]. | ||
| − | |style="text-align:right;"|והרביעית אל השלישית על יחס המוסיף שליש | + | |style="text-align:right;"|והרביעית אל השלישית על {{#annot:sesquitertian|125|R0iD}}יחס המוסיף שליש{{#annotend:R0iD}} |
|- | |- | ||
| | | | ||
*[<math>\scriptstyle{\color{blue}{R_5:R_4}}</math>]: The fifth [line] is in the sesquiquartan ratio to the fourth [line]. | *[<math>\scriptstyle{\color{blue}{R_5:R_4}}</math>]: The fifth [line] is in the sesquiquartan ratio to the fourth [line]. | ||
| − | |style="text-align:right;"|והחמישית אל הרביעית על יחס המוסי' רביע | + | |style="text-align:right;"|והחמישית אל הרביעית על {{#annot:sesquiquartan|125|zpWW}}יחס המוסי' רביע{{#annotend:zpWW}} |
| + | |- | ||
| + | |According to this way the matter proceeds always. | ||
| + | |style="text-align:right;"|ועל הדרך הזה הולך הענין תמיד | ||
| + | |- | ||
| + | |The excess is found as is said: | ||
| + | |style="text-align:right;"|ונמצא ההעדף כפי מה שנאמר לך | ||
|- | |- | ||
| | | | ||
| − | + | *[<math>\scriptstyle R_2-R_1</math>]: The excesses of the second line over the first line differ by number, but not differ by ratio. | |
| − | |||
| − | |||
|style="text-align:right;"|ונמצא תוספת השורה השנית על השורה הראשונה יתחלף במספר ואם לא יתחלף ביחס | |style="text-align:right;"|ונמצא תוספת השורה השנית על השורה הראשונה יתחלף במספר ואם לא יתחלף ביחס | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle{\color{blue}{2-1=1}}</math> | + | :*The excess of the first rubric of [the second line] over the first rubric of the first line is one. <math>\scriptstyle{\color{blue}{2-1=1}}</math> |
|style="text-align:right;"|ונמצא תוספת הבית הראשון ממנו על הבית הראשון מהשורה הראשונה באחד | |style="text-align:right;"|ונמצא תוספת הבית הראשון ממנו על הבית הראשון מהשורה הראשונה באחד | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle{\color{blue}{4-2=2}}</math> | + | :*The excess of the second rubric of [the second line] over the second rubric of the first [line] is two. <math>\scriptstyle{\color{blue}{4-2=2}}</math> |
|style="text-align:right;"|ותוספת הבית השני ממנו על הבית השני מהראשון בשנים | |style="text-align:right;"|ותוספת הבית השני ממנו על הבית השני מהראשון בשנים | ||
|- | |- | ||
| − | | | + | |According to this way by the order of the successive numbers. |
| − | |||
|style="text-align:right;"|ועל הדרך הזה על סדר המספרים הנמשכים | |style="text-align:right;"|ועל הדרך הזה על סדר המספרים הנמשכים | ||
|- | |- | ||
| − | |<math>\scriptstyle | + | | |
| + | :According to this way the property of each rubric to its preceding [<math>\scriptstyle C_mR_{n+1}-C_mR_n=m</math>] | ||
|style="text-align:right;"|ועל הדרך הזה ענין כל בית אצל הקודם אליו‫<ref>marg.א"ב ר"ל שהבית הראשון מהשורה השלישית על הראשון מהשנית יוסיף א' והשני על השני ב' על סדר המספרי' הנמשכים</ref> | |style="text-align:right;"|ועל הדרך הזה ענין כל בית אצל הקודם אליו‫<ref>marg.א"ב ר"ל שהבית הראשון מהשורה השלישית על הראשון מהשנית יוסיף א' והשני על השני ב' על סדר המספרי' הנמשכים</ref> | ||
|- | |- | ||
| − | |<math>\scriptstyle | + | | |
| − | :the | + | *[<math>\scriptstyle C_mR_{n+2}-C_mR_n=2m</math>]: When the third is related to the first in every sequence they are found by the order of the even numbers. |
|style="text-align:right;"|ונמצא זה כאשר יוקש בין השלישי והראשון בכל סדור על סדר הזוגות | |style="text-align:right;"|ונמצא זה כאשר יוקש בין השלישי והראשון בכל סדור על סדר הזוגות | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle{\color{blue}{C_1R_{n+2}-C_1R_n=2}}</math> | + | :*The first [rubric] of each third [line] exceeds by two over the first [rubric] of each first [line]. |
| − | :<math>\scriptstyle{\color{blue}{C_2R_{n+2}-C_2R_n=4}}</math> | + | ::<math>\scriptstyle{\color{blue}{C_1R_{n+2}-C_1R_n=2}}</math> |
| − | :<math>\scriptstyle{\color{blue}{C_3R_{n+2}-C_3R_n=6}}</math> | + | |style="text-align:right;"|כי הנה נמצא הראשון מכל שלישי יוסיף על ראשון מכל ראשון בשנים |
| − | |style="text-align:right;"| | + | |- |
| + | | | ||
| + | :*The second by four. | ||
| + | ::<math>\scriptstyle{\color{blue}{C_2R_{n+2}-C_2R_n=4}}</math> | ||
| + | |style="text-align:right;"|והשני בארבעה | ||
| + | |- | ||
| + | | | ||
| + | :*The third by six. | ||
| + | ::<math>\scriptstyle{\color{blue}{C_3R_{n+2}-C_3R_n=6}}</math> | ||
| + | |style="text-align:right;"|והשלישי בששה | ||
|- | |- | ||
| − | |< | + | | |
| − | :<math>\scriptstyle{\color{blue}{C_1R_{n+3}-C_1R_n=3}}</math | + | :And so on according to this way. |
| + | |style="text-align:right;"|ועל הדרך הזה‫<ref>marg. ר"ל בשורה הראשונה תמצא שכל שלישי לאי זה ראשון שיהיה יוסיף בשנים כמו ג' לא' וד' לב' וה' לג' ובשורה השנית יוסיף בארבעה ובשלישי' בששה</ref> | ||
| + | |- | ||
| + | | | ||
| + | :*The excess of the first rubric of the fourth [line] over the first rubric of each first [line] is three by three. | ||
| + | ::<math>\scriptstyle{\color{blue}{C_1R_{n+3}-C_1R_n=3}}</math> | ||
|style="text-align:right;"|ואמנם תוספת הבית הראשו' מ[הר]ביעי על הבית הראשון מכל ראשון הנה הוא בשלשה שלשה | |style="text-align:right;"|ואמנם תוספת הבית הראשו' מ[הר]ביעי על הבית הראשון מכל ראשון הנה הוא בשלשה שלשה | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle{\color{blue}{C_2R_{n+3}-C_2R_n=6}}</math | + | :*The excess of the second [rubric] of the fourth [line] over the second [rubric] of the first [line] is six by six. |
| + | ::<math>\scriptstyle{\color{blue}{C_2R_{n+3}-C_2R_n=6}}</math> | ||
|style="text-align:right;"|ותוספת השני מן הרביעי על השני מן הראשון בששה ששה | |style="text-align:right;"|ותוספת השני מן הרביעי על השני מן הראשון בששה ששה | ||
|- | |- | ||
| | | | ||
| − | + | *According to this way the excess of each rubric over the [other] rubric on both directions is three by three [<math>\scriptstyle C_mR_{n+3}-C_mR_n=3m</math>] | |
|style="text-align:right;"|ועל הדרך הזה תוספת כל בית יוסיף על תוספת הבית בשני צדדין בשלשה שלשה | |style="text-align:right;"|ועל הדרך הזה תוספת כל בית יוסיף על תוספת הבית בשני צדדין בשלשה שלשה | ||
|- | |- | ||
| − | |<math>\scriptstyle R_4-R_2=2\sdot\left(R_2-R_1\right)</math>? | + | | |
| + | *The excess of the fourth over the second, between them one line, as the excess of the second over the first in ratio [?] | ||
| + | :<math>\scriptstyle R_4-R_2=2\sdot\left(R_2-R_1\right)</math>[?] | ||
|style="text-align:right;"|תוספת רביעי על השני וביניהם שורה אחת כתוספת השני על הראשון ביחס | |style="text-align:right;"|תוספת רביעי על השני וביניהם שורה אחת כתוספת השני על הראשון ביחס | ||
|- | |- | ||
| − | |<math>\scriptstyle R_6-R_3=3\sdot\left(R_2-R_1\right)</math>? | + | | |
| + | *The excess of the sixth over the third, between them two lines, as the excess of the third over the second in ratio [?] | ||
| + | :<math>\scriptstyle R_6-R_3=3\sdot\left(R_2-R_1\right)</math>[?] | ||
|style="text-align:right;"|ותוספת הששי על השלישי וביניהם שתי שורות כתוספת השלישי על השני ביחס ועל הדרך הזה‫<ref>marg. א"ב צ"ע בזה שורה אחת כי יש טעות בלשון ובסברא אפשר לכוין הלשון על צדדים רבים ולכן לא תקננו כי לא יודע אל איזה כיון המחבר</ref> | |style="text-align:right;"|ותוספת הששי על השלישי וביניהם שתי שורות כתוספת השלישי על השני ביחס ועל הדרך הזה‫<ref>marg. א"ב צ"ע בזה שורה אחת כי יש טעות בלשון ובסברא אפשר לכוין הלשון על צדדים רבים ולכן לא תקננו כי לא יודע אל איזה כיון המחבר</ref> | ||
|- | |- | ||
| − | | | + | | |
| + | *Every number on the principal diagonal is a square. | ||
|style="text-align:right;"|והנה תמצא כל מספר ממספרי הקטר מרבע | |style="text-align:right;"|והנה תמצא כל מספר ממספרי הקטר מרבע | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle{\color{blue}{4;\ 9;\ 16}}</math> | + | ::Such as: <math>\scriptstyle{\color{blue}{4;\ 9;\ 16}}</math> |
|style="text-align:right;"|כמו הד' והט' והי"ו | |style="text-align:right;"|כמו הד' והט' והי"ו | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"|ונמצא מקבץ כל שני מרבעים נמשכים ומקבץ השני שטחים אשר יפלו | + | *The sum of every two consecutive square numbers plus the numbers on their inverse diagonal is a square number. |
| + | |style="text-align:right;"|ונמצא מקבץ כל שני מרבעים נמשכים ומקבץ השני שטחים אשר יפלו ביניהם על האלכסוניות מרבע | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle{\color{blue}{\left(4+9\right)+\left(6+6\right)=25 | + | ::Such as: <math>\scriptstyle{\color{blue}{\left(4+9\right)+\left(6+6\right)=25}}</math> |
|style="text-align:right;"|כמו מקבץ הד' והט' עם הו' וו' שעולה כ"ה | |style="text-align:right;"|כמו מקבץ הד' והט' עם הו' וו' שעולה כ"ה | ||
|- | |- | ||
| − | | | + | | |
| + | *The sum of every two consecutive square numbers exceeds the sum of the numbers on their inverse diagonal by one. | ||
|style="text-align:right;"|ונמצא מקבץ כל שני מרבעים נמשכים על מקבץ השני שטחים מוסיף באחד | |style="text-align:right;"|ונמצא מקבץ כל שני מרבעים נמשכים על מקבץ השני שטחים מוסיף באחד | ||
|- | |- | ||
| − | |<math>\scriptstyle\left[2\sdot\left[n^2+\left(n+1\right)^2\right]\right]-1=m^2</math | + | | |
| + | *Double the sum of every two consecutive square numbers minus one is necessarily a square number. | ||
| + | :<math>\scriptstyle\left[2\sdot\left[n^2+\left(n+1\right)^2\right]\right]-1=m^2</math> | ||
|style="text-align:right;"|והנה יחויב שיהיה כפל מקובץ כל שני מרבעים נמשכים מחוסר ממנו אחד מרבע‫<ref>marg.לפי שמקובץ כפל השטחים מרבע בתוספת אחד</ref><ref>marg.כמו כפל ד"ט שהוא כ"ו וכ"ה מרבע</ref> | |style="text-align:right;"|והנה יחויב שיהיה כפל מקובץ כל שני מרבעים נמשכים מחוסר ממנו אחד מרבע‫<ref>marg.לפי שמקובץ כפל השטחים מרבע בתוספת אחד</ref><ref>marg.כמו כפל ד"ט שהוא כ"ו וכ"ה מרבע</ref> | ||
|- | |- | ||
| − | |<math>\scriptstyle R_nC_m\times R_aC_b=R_aC_m\times R_nC_b</math> | + | | |
| + | *The product of every number in a line by a number in another line is equal to the product of the corresponding by the corresponding. | ||
| + | :<math>\scriptstyle R_nC_m\times R_aC_b=R_aC_m\times R_nC_b</math> | ||
|style="text-align:right;"|ונמצא הכאת כל מספר משורה במספר משורה אחרת שוה להכאת בן גילו בבן גילו | |style="text-align:right;"|ונמצא הכאת כל מספר משורה במספר משורה אחרת שוה להכאת בן גילו בבן גילו | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle{\color{blue}{R_1C_2\times R_2C_{10}=R_2C_2\times R_1C_{10} | + | ::Such as: 2, which is the second in the first [line], by 20, which is the last of the second [line], that is the same as 4, which is the second in the second [line], by 10, which is the last of the first [line] |
| − | + | ::<math>\scriptstyle{\color{blue}{R_1C_2\times R_2C_{10}=2\sdot20=4\sdot10=R_2C_2\times R_1C_{10}}}</math> | |
|style="text-align:right;"|כמו הב' והוא השני מן הראשונה בכ' והוא האחרון מן השנית אשר הוא כמו הד' אשר הוא השני מן השנית בי' אשר הוא האחרון מן הראשונה | |style="text-align:right;"|כמו הב' והוא השני מן הראשונה בכ' והוא האחרון מן השנית אשר הוא כמו הד' אשר הוא השני מן השנית בי' אשר הוא האחרון מן הראשונה | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"|ונמצא הכאת כל מספר ממספרי הקטר בבן גילו מהצד | + | *The product of every number on the principal diagonal by its inverse number on the other side of the principal diagonal is equal to the product of the corresponding numbers by each other, meaning on the inverse diagonal. |
| + | |style="text-align:right;"|ונמצא הכאת כל מספר ממספרי הקטר בבן גילו מהצד האחר מזה הקטר כמו בני גילם אחד מהם באחר רצוני מן הקטר האחר | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle{\color{blue}{1\sdot100=10\sdot10}}</math | + | ::Such as: <math>\scriptstyle{\color{blue}{1\sdot100=10\sdot10}}</math> |
|style="text-align:right;"|כמו הכאת הא' בק' אשר הוא כמו הכאת הי' בי‫' | |style="text-align:right;"|כמו הכאת הא' בק' אשר הוא כמו הכאת הי' בי‫' | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle{\color{blue}{4\sdot81=18\sdot18}}</math | + | ::Also: <math>\scriptstyle{\color{blue}{4\sdot81=18\sdot18}}</math> |
| − | |style="text-align:right;"|וג"כ הכאת ד' בפ"א אשר הוא כמו הכאת י"ח בי"ח | + | |style="text-align:right;"|וג"כ הכאת ד' בפ"א אשר הוא כמו הכאת י"ח בי"ח |
|- | |- | ||
| | | | ||
| + | ::And so on by this way. | ||
| + | |style="text-align:right;"|ועל הדרך הזה | ||
| + | |- | ||
| + | |As for the other ratios, before examining them in this table, the quality of rule of investigating their first numbers will be referred to. | ||
|style="text-align:right;"|ואמנם היחסים האחרים הנה אנחנו קודם שנבחין אותם מזה הלוח הבעל טורים נרמוז אל איכות ההנהגה בדרישת מספריהם הראשונים | |style="text-align:right;"|ואמנם היחסים האחרים הנה אנחנו קודם שנבחין אותם מזה הלוח הבעל טורים נרמוז אל איכות ההנהגה בדרישת מספריהם הראשונים | ||
|- | |- | ||
| − | | | + | |Their properties will be determined, then their examination in this table will be alluded to. |
|style="text-align:right;"|ונרמוז אל ענינים מסגלים להם אח"כ נרמוז אל בחינתם מזה הלוח | |style="text-align:right;"|ונרמוז אל ענינים מסגלים להם אח"כ נרמוז אל בחינתם מזה הלוח | ||
| + | |} | ||
| + | {| | ||
|- | |- | ||
| | | | ||
| Line 2,028: | Line 2,415: | ||
| | | | ||
|- | |- | ||
| − | | | + | |The superbipartient ratio or the superpartient ratio is sometimes absolute and sometimes not absolute. |
| − | |style="text-align:right;"|ונאמר אם יחס המוסיף בשני חלקים או המוסיף בחלקים הנה לפעמים יהיה גמור ולפעמים לא יהיה גמור | + | |style="text-align:right;"|ונאמר אם {{#annot:superbipartient|128|ppa4}}יחס המוסיף בשני חלקים{{#annotend:ppa4}} או {{#annot:term|1660,128|G6ks}}המוסיף בחלקים{{#annotend:G6ks}} הנה לפעמים יהיה גמור ולפעמים לא יהיה גמור |
|- | |- | ||
| − | | | + | |The absolute, meaning that which is not reduced to the superparticular ratio. |
|style="text-align:right;"|והגמור רצוני מה שאיננו חוזר אל יחס דמיון וחלק | |style="text-align:right;"|והגמור רצוני מה שאיננו חוזר אל יחס דמיון וחלק | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle{\color{blue}{1:\left(1+\frac{2}{6}\right)=1:\left(1+\frac{1}{3}\right)}}</math> | + | :Such as: |
| + | :*The superbisextan which is reduced to the sesquitertian. | ||
| + | ::<math>\scriptstyle{\color{blue}{1:\left(1+\frac{2}{6}\right)=1:\left(1+\frac{1}{3}\right)}}</math> | ||
|style="text-align:right;"|כמו חזרת המוסיף בשני ששיות אל המוסיף שליש | |style="text-align:right;"|כמו חזרת המוסיף בשני ששיות אל המוסיף שליש | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle{\color{blue}{1:\left(1+\frac{2}{4}\right)=1:\left(1+\frac{1}{2}\right)}}</math> | + | :*The superbiquartan which is reduced to the sesquialter. |
| + | ::<math>\scriptstyle{\color{blue}{1:\left(1+\frac{2}{4}\right)=1:\left(1+\frac{1}{2}\right)}}</math> | ||
|style="text-align:right;"|והמוסיף בשני רביעיות אל המוסיף חצי | |style="text-align:right;"|והמוסיף בשני רביעיות אל המוסיף חצי | ||
|- | |- | ||
| | | | ||
| − | <math>\scriptstyle1:\left(1+\frac{2}{2n}\right)=1:\left(1+\frac{1}{n}\right)</math> | + | *Every superbipartient that is denominated by an even number [is reducible]. |
| − | |style="text-align:right;"|כל מוסיף בשני חלקים נקראים בשם זוג | + | :[<math>\scriptstyle1:\left(1+\frac{2}{2n}\right)=1:\left(1+\frac{1}{n}\right)</math>] |
| + | |style="text-align:right;"|כל {{#annot:superbipartient|128|bySx}}מוסיף בשני חלקים{{#annotend:bySx}} נקראים בשם זוג | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle{\color{blue}{1:\left(1+\frac{3}{6}\right)=1:\left(1+\frac{1}{2}\right)}}</math> | + | :*The supertrisextan is reduced to the sesquialter. |
| + | ::<math>\scriptstyle{\color{blue}{1:\left(1+\frac{3}{6}\right)=1:\left(1+\frac{1}{2}\right)}}</math> | ||
|style="text-align:right;"|וחזרת המוסיף בשלשה ששיות אל החצי אחר כן | |style="text-align:right;"|וחזרת המוסיף בשלשה ששיות אל החצי אחר כן | ||
|- | |- | ||
| − | | | + | |Such as the superbiquintan <math>\scriptstyle{\color{blue}{1:\left(1+\frac{2}{5}\right)}}</math> and the supertriquartan <math>\scriptstyle{\color{blue}{1:\left(1+\frac{3}{4}\right)}}</math> are the [absolute ratios]. |
| − | + | |style="text-align:right;"|ואבל כמו {{#annot:superbiquintan|128|9hYQ}}המוסיף בשני חמישיות{{#annotend:9hYQ}} ו{{#annot:supertriquartan|128|IWG3}}המוסיף בשלש רביעיות{{#annotend:IWG3}} הוא הבלתי גמור | |
| − | |||
| − | |style="text-align:right;"|ואבל כמו המוסיף בשני חמישיות | ||
|- | |- | ||
| − | | | + | |There is no common order for the absolute ratios, thus any one who measures should examine the sequence. |
|style="text-align:right;"|ולא ימצא לגמור סדור משותף בו אבל יצטרך כל שוער להבחין הסדור | |style="text-align:right;"|ולא ימצא לגמור סדור משותף בו אבל יצטרך כל שוער להבחין הסדור | ||
|- | |- | ||
| − | |<math>\scriptstyle1:\left(1+\frac{m}{n}\right)=n:\left(n+m\right)</math> | + | |When it is taken in actu, the order of the realization of its first number is such that the first is that by which the part of the numbers is denominated, so that two is added to it if there are two parts or three if there are three parts. |
| + | :[<math>\scriptstyle1:\left(1+\frac{m}{n}\right)=n:\left(n+m\right)</math>] | ||
|style="text-align:right;"|ואמנם כאשר ילקח בשלוח‫<ref>marg.ר"ל מבלי בחינה בין הגמור והבלתי גמור</ref> הנה הסדר בהגעת מספרו הראשון הוא שיגיע הראשון אשר בשמו יקרא זה החלק מן המספרי' כדי שנוסיף עליו אם הוא שני חלקים הנה שנים ואם היה שלשה חלקים הנה השלשה | |style="text-align:right;"|ואמנם כאשר ילקח בשלוח‫<ref>marg.ר"ל מבלי בחינה בין הגמור והבלתי גמור</ref> הנה הסדר בהגעת מספרו הראשון הוא שיגיע הראשון אשר בשמו יקרא זה החלק מן המספרי' כדי שנוסיף עליו אם הוא שני חלקים הנה שנים ואם היה שלשה חלקים הנה השלשה | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle{\color{blue}{1:\left(1+\frac{2}{3}\right)=3:\left(3+2\right)=3:5}}</math> | + | ::For example: |
| + | ::*If the addition is of two thirds [superbitertian], the three are set, then two are added to them, which are five, so their beginning is three and five. | ||
| + | :::[<math>\scriptstyle{\color{blue}{1:\left(1+\frac{2}{3}\right)=3:\left(3+2\right)=3:5}}</math>] | ||
|style="text-align:right;"|המשל בזה אם היה התוספת בשני שלישיות נניח שלשה ונוסיף עליהם שנים ויהיו חמשה ותהיה התחלתם משלשה וחמשה | |style="text-align:right;"|המשל בזה אם היה התוספת בשני שלישיות נניח שלשה ונוסיף עליהם שנים ויהיו חמשה ותהיה התחלתם משלשה וחמשה | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle{\color{blue}{1:\left(1+\frac{3}{4}\right)=4:\left(4+3\right)=4:7}}</math> | + | ::*If the addition is of three quarters [supertriquartan], the four are set, then three are added to them, and they are four and seven that are their beginning. |
| + | :::[<math>\scriptstyle{\color{blue}{1:\left(1+\frac{3}{4}\right)=4:\left(4+3\right)=4:7}}</math>] | ||
|style="text-align:right;"|ואם יהיה התוספת בשלשה רביעיות נניח ארבעה ונוסיף עליהם שלשה ויהיו ארבעה ושבעה והיא מתחלתם | |style="text-align:right;"|ואם יהיה התוספת בשלשה רביעיות נניח ארבעה ונוסיף עליהם שלשה ויהיו ארבעה ושבעה והיא מתחלתם | ||
|- | |- | ||
| − | |<math>\scriptstyle1:\left(1+\frac{2}{n}\right)</math> | + | | |
| + | *Finding the smaller numbers in the superbipartient ratios <math>\scriptstyle1:\left(1+\frac{2}{n}\right)</math>: | ||
|style="text-align:right;"|והנה תמצא המספרים החסרים ביחס הדמיון ושני חלקים | |style="text-align:right;"|והנה תמצא המספרים החסרים ביחס הדמיון ושני חלקים | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle1:\left(1+\frac{2}{3}\right)</math> | + | :*Superbitertian ratio <math>\scriptstyle1:\left(1+\frac{2}{3}\right)</math> |
| − | |style="text-align:right;"|אם | + | |style="text-align:right;"|אם ביחס הדמיון ושני שלישיות |
|- | |- | ||
| | | | ||
| − | ::less | + | ::*the less are added three by three |
|style="text-align:right;"|הנה החסרים מוסיפים בשלשה | |style="text-align:right;"|הנה החסרים מוסיפים בשלשה | ||
|- | |- | ||
| | | | ||
| − | ::greater | + | ::*the greater [are added] five by five |
|style="text-align:right;"|והנוספים בחמשה | |style="text-align:right;"|והנוספים בחמשה | ||
|- | |- | ||
| Line 2,089: | Line 2,484: | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle1:\left(1+\frac{2}{4}\right)</math> | + | :*Superbiquartan ratio <math>\scriptstyle1:\left(1+\frac{2}{4}\right)</math>, which is not absolute |
| − | |style="text-align:right;"|ואמנם | + | |style="text-align:right;"|ואמנם ביחס הדמיון ושתי רביעיות והוא בלתי גמור |
|- | |- | ||
| | | | ||
| − | ::less | + | ::*the less are added four by four |
|style="text-align:right;"|הנה תמצא החסרים יוסיפו בארבעה ארבעה | |style="text-align:right;"|הנה תמצא החסרים יוסיפו בארבעה ארבעה | ||
|- | |- | ||
| | | | ||
| − | ::greater | + | ::*the greater [are added] six by six |
|style="text-align:right;"|והנוספים בששה ששה | |style="text-align:right;"|והנוספים בששה ששה | ||
|- | |- | ||
| Line 2,105: | Line 2,500: | ||
|- | |- | ||
| | | | ||
| + | :According to this way the less are by themselves and the greater are by themselves. | ||
|style="text-align:right;"|ועל הדרך הזה החסר כמו עצמו והנוסף כמו עצמו | |style="text-align:right;"|ועל הדרך הזה החסר כמו עצמו והנוסף כמו עצמו | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle1:\left(1+\frac{2}{5}\right)</math> | + | :*By this order, the superbiquintan <math>\scriptstyle1:\left(1+\frac{2}{5}\right)</math> |
|style="text-align:right;"|וכפי זה הוא הסדר במוסיף שתי חמישיות | |style="text-align:right;"|וכפי זה הוא הסדר במוסיף שתי חמישיות | ||
|- | |- | ||
| − | |<math>\scriptstyle\left[1:\left(1+\frac{2}{n}\right)\right]:\left[1:\left(1+\frac{2}{n+1}\right)\right]</math> | + | |Comparing [the superbipartient ratios] to each other [<math>\scriptstyle\left[1:\left(1+\frac{2}{n}\right)\right]:\left[1:\left(1+\frac{2}{n+1}\right)\right]</math>]: |
|style="text-align:right;"|ואמנם הקש קצתם אל קצת | |style="text-align:right;"|ואמנם הקש קצתם אל קצת | ||
|- | |- | ||
| | | | ||
| + | :i.e. comparing the superbitertian to the superbiquartan, then to the superbiquintan | ||
:<math>\scriptstyle\left[1:\left(1+\frac{2}{3}\right)\right];\quad\left[1:\left(1+\frac{2}{4}\right)\right];\quad\left[1:\left(1+\frac{2}{5}\right)\right]</math> | :<math>\scriptstyle\left[1:\left(1+\frac{2}{3}\right)\right];\quad\left[1:\left(1+\frac{2}{4}\right)\right];\quad\left[1:\left(1+\frac{2}{5}\right)\right]</math> | ||
| − | |style="text-align:right;"|רצוני הקש המוסיף שתי שלישיות אל המוסיף שתי רביעיות אח"כ המוסיף שתי חמישיות | + | |style="text-align:right;"|רצוני הקש {{#annot:superbitertian|128|zbbR}}המוסיף שתי שלישיות{{#annotend:zbbR}} אל המוסיף שתי רביעיות אח"כ {{#annot:superbiquintan|128|Exgb}}המוסיף שתי חמישיות{{#annotend:Exgb}} |
|- | |- | ||
| − | |less | + | | |
| + | :*the less are added one by one. | ||
|style="text-align:right;"|הנה החסרים יוסיפו באחד אחד | |style="text-align:right;"|הנה החסרים יוסיפו באחד אחד | ||
|- | |- | ||
| − | |greater | + | | |
| − | |style="text-align:right;"|והנוספים ג"כ יוסיפו באחד אחד‫<ref>marg. א"ב לפי שמדרגתם ג"ה ד"ו ה"ז וג"ה יתוסף | + | :*the greater are also added one by one. |
| + | |style="text-align:right;"|והנוספים ג"כ יוסיפו באחד אחד‫<ref>marg. א"ב לפי שמדרגתם ג"ה ד"ו ה"ז וג"ה יתוסף תמיד בג"ה וד"ו בד"ו וה"ז בה"ז הנה תוספת החסרים והנוספי' אינו רק באחד אחד</ref> | ||
|- | |- | ||
| − | |<math>\scriptstyle\left[1:\left(1+\frac{2}{2n-1}\right)\right]:\left[1:\left(1+\frac{2}{2n+1}\right)\right]</math> | + | | |
| + | *When examine the absolute [superbipartient] ratios, they are by the sequence of the successive odd numbers. | ||
| + | :[<math>\scriptstyle\left[1:\left(1+\frac{2}{2n-1}\right)\right]:\left[1:\left(1+\frac{2}{2n+1}\right)\right]</math>] | ||
|style="text-align:right;"|וכאשר תבחין הגמורים בזה היחס יהיו על סדר הנפרדים הנמשכים | |style="text-align:right;"|וכאשר תבחין הגמורים בזה היחס יהיו על סדר הנפרדים הנמשכים | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle1:\left(1+\frac{2}{3}\right)\longrightarrow{\color{blue}{3:5}}</math> | + | ::Such as: |
| − | |style="text-align:right;"|כמו החמשה אל השלשה והוא | + | ::*five to three, which is the superbitertian <math>\scriptstyle1:\left(1+\frac{2}{3}\right)\longrightarrow{\color{blue}{3:5}}</math> |
| + | |style="text-align:right;"|כמו החמשה אל השלשה והוא {{#annot:superbitertian|128|fH6C}}המוסיף בשתי שלישיות{{#annotend:fH6C}} | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle1:\left(1+\frac{2}{5}\right)\longrightarrow{\color{blue}{5:7}}</math> | + | ::*seven to five, which is the superbiquintan <math>\scriptstyle1:\left(1+\frac{2}{5}\right)\longrightarrow{\color{blue}{5:7}}</math> |
| − | |style="text-align:right;"|והשבעה אל החמשה והוא | + | |style="text-align:right;"|והשבעה אל החמשה והוא {{#annot:superbiquintan|128|w5sR}}המוסיף בשתי חמישיות{{#annotend:w5sR}} |
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle1:\left(1+\frac{2}{7}\right)\longrightarrow{\color{blue}{7:9}}</math> | + | ::*nine to seven, which is the superbiseptian <math>\scriptstyle1:\left(1+\frac{2}{7}\right)\longrightarrow{\color{blue}{7:9}}</math> |
| − | |style="text-align:right;"|והתשעה אל השבעה והוא | + | |style="text-align:right;"|והתשעה אל השבעה והוא המוסיף בשתי שביעיות |
|- | |- | ||
| − | |<math>\scriptstyle1:\left(1+\frac{m}{n}\right)</math> | + | |Comparing between the supermultiplepartient [<math>\scriptstyle1:\left(1+\frac{m}{n}\right)</math>]: |
|style="text-align:right;"|ואמנם ההקש בין רבי החלקים | |style="text-align:right;"|ואמנם ההקש בין רבי החלקים | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle1:\left(1+\frac{3}{4}\right)</math> | + | :Such as: |
| − | |style="text-align:right;"|כמו המוסיף בדמיונו ושלשה רביעיות הנה יוסיפו החסרים מהם והמוסיפים מהם על ההקש הנזכר | + | :*the supertriquartan <math>\scriptstyle1:\left(1+\frac{3}{4}\right)</math> |
| + | |style="text-align:right;"|כמו המוסיף בדמיונו ושלשה רביעיות | ||
| + | |- | ||
| + | | | ||
| + | :Their less and their greater are added by the mentioned rule. | ||
| + | |style="text-align:right;"|הנה יוסיפו החסרים מהם והמוסיפים מהם על ההקש הנזכר | ||
|- | |- | ||
| | | | ||
| Line 2,151: | Line 2,558: | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle1:\left(1+\frac{3}{5}\right)</math> | + | :*By this way the addition of three fifthes [supertriquintan] <math>\scriptstyle1:\left(1+\frac{3}{5}\right)</math> |
|style="text-align:right;"|ועל הדרך הזה תוספת שלש חמישיות | |style="text-align:right;"|ועל הדרך הזה תוספת שלש חמישיות | ||
|- | |- | ||
| Line 2,159: | Line 2,566: | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle\left[1:\left(1+\frac{3}{4}\right)\right];\quad\left[1:\left(1+\frac{3}{5}\right)\right];\quad\left[1:\left(1+\frac{3}{6}\right)\right]</math> | + | :The relation between the [supertripartient ratios] is as said for the former [= the superbipartient ratios] |
| − | + | :[<math>\scriptstyle\left[1:\left(1+\frac{3}{4}\right)\right];\quad\left[1:\left(1+\frac{3}{5}\right)\right];\quad\left[1:\left(1+\frac{3}{6}\right)\right]</math>] | |
|style="text-align:right;"|ויהיה התיחסות מה שביניהם כפי מה שנאמר בראשונים | |style="text-align:right;"|ויהיה התיחסות מה שביניהם כפי מה שנאמר בראשונים | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle{\color{blue}{4:7;\quad 5:8;\quad 6:9}}</math> | + | ::Such as: <math>\scriptstyle{\color{blue}{4:7;\quad 5:8;\quad 6:9}}</math> |
|style="text-align:right;"|כמו ארבעה ושבעה אח"כ חמשה ושמנה אח"כ ששה ותשעה | |style="text-align:right;"|כמו ארבעה ושבעה אח"כ חמשה ושמנה אח"כ ששה ותשעה | ||
|- | |- | ||
| − | | | + | |The absolute ratios have unordered properties that should be drawn by examination for each category. |
|style="text-align:right;"|וימצאו לגמורי' סגלות בלתי מסודרות אלא בשער שער יש להוציא אותם עם הבחינה | |style="text-align:right;"|וימצאו לגמורי' סגלות בלתי מסודרות אלא בשער שער יש להוציא אותם עם הבחינה | ||
| + | |} | ||
| + | {| | ||
|- | |- | ||
| | | | ||
| Line 2,181: | Line 2,590: | ||
| | | | ||
|- | |- | ||
| − | | | + | |When wishing to find the first number in the [multiple] superparticular ratio: |
|style="text-align:right;"|וכאשר נרצה שנמצא ראשון המספרים ליחס הדמיון והחלק‫<ref>marg. א"ב צ"ע כי כפי המשך המשלים נראה שהנכון שיאמר הדמיונים והחלק או הכפל והחלק</ref> | |style="text-align:right;"|וכאשר נרצה שנמצא ראשון המספרים ליחס הדמיון והחלק‫<ref>marg. א"ב צ"ע כי כפי המשך המשלים נראה שהנכון שיאמר הדמיונים והחלק או הכפל והחלק</ref> | ||
|- | |- | ||
| − | |<math>\scriptstyle1:\left(2+\frac{1}{n}\right)=n:\left(2n+1\right)</math> | + | |Taking that by which the parts are denominated, as two for a half, three for a third, then multiplying this number by two and adding one to it. |
| + | :<math>\scriptstyle1:\left(2+\frac{1}{n}\right)=n:\left(2n+1\right)</math> | ||
|style="text-align:right;"|הנה נקח אשר על שמו יקראו החלקים כמו השנים לחצי והשלשה לשליש ונכפול זה המספר בשנים ונוסיף עליו אחד | |style="text-align:right;"|הנה נקח אשר על שמו יקראו החלקים כמו השנים לחצי והשלשה לשליש ונכפול זה המספר בשנים ונוסיף עליו אחד | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle{\color{blue}{1:\left(2+\frac{1}{2}\right)=2:\left[\left(2\sdot2\right)+1\right]=2:5}}</math> | + | :Such as: |
| − | |style="text-align:right;"|כמו | + | :*The double sesquialter, for its production is by multiplying the two by two and adding one to it, so they are two and five. |
| + | ::[<math>\scriptstyle{\color{blue}{1:\left(2+\frac{1}{2}\right)=2:\left[\left(2\sdot2\right)+1\right]=2:5}}</math>] | ||
| + | |style="text-align:right;"|כמו הכפל וחצי כי התילדותו מכפל השנים בשנים והתוספת אחד עליו ויהיו שנים וחמשה | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle{\color{blue}{1:\left(2+\frac{1}{3}\right)=3:\left[\left(2\sdot3\right)+1\right]=3:7}}</math> | + | :*The double sesquitertian, whose production is by multiplying the three by two and adding one to it, so they are three and seven. |
| − | |style="text-align:right;"| | + | ::[<math>\scriptstyle{\color{blue}{1:\left(2+\frac{1}{3}\right)=3:\left[\left(2\sdot3\right)+1\right]=3:7}}</math>] |
| + | |style="text-align:right;"|והכפל ושליש שהתילדותו מכפילת השלשה בשנים והתוספת אחד עליו ויהיו שלשה ושבעה | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle{\color{blue}{1:\left(2+\frac{1}{4}\right)=4:\left[\left(2\sdot4\right)+1\right]=4:9}}</math> | + | :*The double sesquiquartan - they are four and nine |
| − | |style="text-align:right;"| | + | ::[<math>\scriptstyle{\color{blue}{1:\left(2+\frac{1}{4}\right)=4:\left[\left(2\sdot4\right)+1\right]=4:9}}</math>] |
| + | |style="text-align:right;"|והכפל ורביע שיהיו ארבעה ותשעה | ||
|- | |- | ||
| | | | ||
| − | + | *Finding the numbers of the first [= the double sesquialter ratio <math>\scriptstyle1:\left(2+\frac{1}{2}\right)</math>]: | |
| − | : | + | |style="text-align:right;"|ונמצאו המספרים בראשון‫<ref>marg. ר"ל ביחס הכפל וחצי</ref> |
| − | |style="text-align:right;"|ונמצאו המספרים בראשון<ref>marg. ר"ל ביחס הכפל וחצי</ref> | ||
|- | |- | ||
| | | | ||
| − | ::greater | + | :*The less are added two by two, by the sequence of the successive even numbers |
| + | |style="text-align:right;"|יתוסף החסר בשנים שנים על סדר הזוגות הנמשכים | ||
| + | |- | ||
| + | | | ||
| + | :*The greater are added five by five. | ||
|style="text-align:right;"|ויתוסף המוסיף בחמשה חמשה | |style="text-align:right;"|ויתוסף המוסיף בחמשה חמשה | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{2:5;\quad 4:10;\quad 6:15}}</math> | + | ::So they are in the double sesquialter: <math>\scriptstyle{\color{blue}{2:5;\quad 4:10;\quad 6:15}}</math> |
|style="text-align:right;"|עד שיהיה במוסיף כפל וחצי שנים וחמשה אח"כ ארבעה ועשרה אח"כ ששה וחמשה עשר | |style="text-align:right;"|עד שיהיה במוסיף כפל וחצי שנים וחמשה אח"כ ארבעה ועשרה אח"כ ששה וחמשה עשר | ||
|- | |- | ||
| | | | ||
| − | + | *Finding the numbers of the second, which is the double sesquitertian ratio <math>\scriptstyle1:\left(2+\frac{1}{3}\right)</math>: | |
| − | : | + | |style="text-align:right;"|ונמצא המספרים בשני והוא יחס הכפל והשליש |
| − | |style="text-align:right;"|ונמצא המספרים בשני והוא | + | |- |
| + | | | ||
| + | :*The less are added three by three. | ||
| + | |style="text-align:right;"|יתוספו החסרים בהם בשלשה שלשה | ||
|- | |- | ||
| | | | ||
| − | : | + | :*The greater [are added] seven by seven. |
|style="text-align:right;"|והמוסיפים בשבעה שבעה | |style="text-align:right;"|והמוסיפים בשבעה שבעה | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{3:7;\quad 6:14;\quad 9:21}}</math> | + | ::Such as: <math>\scriptstyle{\color{blue}{3:7;\quad 6:14;\quad 9:21}}</math> |
|style="text-align:right;"|כמו שלשה ושבעה אח"כ ששה וארבעה עשר ותשעה ואחד ועשרים | |style="text-align:right;"|כמו שלשה ושבעה אח"כ ששה וארבעה עשר ותשעה ואחד ועשרים | ||
|- | |- | ||
| | | | ||
| − | + | *Finding the numbers of the third, which is the double sesquiquartan ratio <math>\scriptstyle1:\left(2+\frac{1}{4}\right)</math>: | |
| − | + | |style="text-align:right;"|ונמצאו המספרים בשלישי והוא יחס הכפל ורביע | |
| − | |style="text-align:right;"|ונמצאו המספרים בשלישי והוא | ||
|- | |- | ||
| | | | ||
| − | ::greater | + | :*The less are four by four |
| + | |style="text-align:right;"|יתוסף החסר בהם בארבעה ארבעה | ||
| + | |- | ||
| + | | | ||
| + | :*The greater [are] nine by nine | ||
|style="text-align:right;"|והמוסיף בתשעה תשעה | |style="text-align:right;"|והמוסיף בתשעה תשעה | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{4:9;\quad 8:18;\quad 12:27}}</math> | + | ::So they are according to the succession of: <math>\scriptstyle{\color{blue}{4:9;\quad 8:18;\quad 12:27}}</math> |
|style="text-align:right;"|עד שיהיו על משך ארבעה ותשעה אח"כ שמנה ושמנה עשר אח"כ שנים עשר ושבעה ועשרים | |style="text-align:right;"|עד שיהיו על משך ארבעה ותשעה אח"כ שמנה ושמנה עשר אח"כ שנים עשר ושבעה ועשרים | ||
|- | |- | ||
| − | | | + | |As a rule: the addition of the less and the greater is according to their first number. |
|style="text-align:right;"|ובכלל הנה תוספת החסר והנוסף יהיה על מספרם הראשון | |style="text-align:right;"|ובכלל הנה תוספת החסר והנוסף יהיה על מספרם הראשון | ||
|- | |- | ||
| − | |<math>\scriptstyle\left[1:\left(2+\frac{1}{n}\right)\right]:\left[1:\left(2+\frac{1}{n+1}\right)\right]</math> | + | |The relation between their terms, meaning between the double sesquialter ratio and the double sesquitertian ratio [and the double sesquiquartan ratio] [<math>\scriptstyle\left[1:\left(2+\frac{1}{n}\right)\right]:\left[1:\left(2+\frac{1}{n+1}\right)\right]</math>] |
| − | :<math>\scriptstyle\left[1:\left(2+\frac{1}{2}\right)\right];\quad\left[1:\left(2+\frac{1}{3}\right)\right];\quad\left[1:\left(2+\frac{1}{4}\right)\right]</math> | + | :[<math>\scriptstyle\left[1:\left(2+\frac{1}{2}\right)\right];\quad\left[1:\left(2+\frac{1}{3}\right)\right];\quad\left[1:\left(2+\frac{1}{4}\right)\right]</math>] |
|style="text-align:right;"|ואמנם ההתיחסות במה שבין מדרגותיהם רצוני התיחסות מה שבין הכפל והחצי ובין הכפל והשליש | |style="text-align:right;"|ואמנם ההתיחסות במה שבין מדרגותיהם רצוני התיחסות מה שבין הכפל והחצי ובין הכפל והשליש | ||
|- | |- | ||
| − | |less | + | | |
| + | *The less are added one by one. | ||
|style="text-align:right;"|הנה החסרים יתוספו באחד אחד | |style="text-align:right;"|הנה החסרים יתוספו באחד אחד | ||
|- | |- | ||
| − | |greater | + | | |
| + | *The greater are added two by two, according to the multiple [n+2] | ||
|style="text-align:right;"|והמוסיפים בשנים שנים כפי ההכפלה | |style="text-align:right;"|והמוסיפים בשנים שנים כפי ההכפלה | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle{\color{blue}{2:5;\quad 3:7;\quad 4:9}}</math> | + | :So they are: <math>\scriptstyle{\color{blue}{2:5;\quad 3:7;\quad 4:9}}</math> and so on in this way. |
|style="text-align:right;"|עד שיהיו שנים וחמשה שלשה ושבעה ארבעה ותשעה ועל הדרך הזה | |style="text-align:right;"|עד שיהיו שנים וחמשה שלשה ושבעה ארבעה ותשעה ועל הדרך הזה | ||
|- | |- | ||
| − | |odd numbers | + | |Thus, the rule of the addition follows the successive odd numbers. |
|style="text-align:right;"|וילך מנהג התוספת על הנפרדים הנמשכים | |style="text-align:right;"|וילך מנהג התוספת על הנפרדים הנמשכים | ||
| + | |} | ||
| + | {| | ||
|- | |- | ||
| | | | ||
| Line 2,265: | Line 2,692: | ||
|- | |- | ||
| | | | ||
| + | *The double [superbipartient] ratios - their production should be done as was already done [in the multiple superparticular ratios], except adding two instead of one. | ||
|style="text-align:right;"|ואמנם יחסי הכפל והשני שלישיות הנה ראוי שנעשה בתולדתם מה שכבר עשינו אותו אלא שאנחנו נוסיף תמורת האחד שנים | |style="text-align:right;"|ואמנם יחסי הכפל והשני שלישיות הנה ראוי שנעשה בתולדתם מה שכבר עשינו אותו אלא שאנחנו נוסיף תמורת האחד שנים | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle1:\left(2+\frac{2}{3}\right)\longrightarrow{\color{blue}{3:8}}</math> | + | :*The double superbitertian ratio - starting from three and eight. |
| + | ::<math>\scriptstyle1:\left(2+\frac{2}{3}\right)\longrightarrow{\color{blue}{3:8}}</math> | ||
|style="text-align:right;"|ונתחיל אם ביחס הכפל והשתי שלישיות מן השלשה והשמנה | |style="text-align:right;"|ונתחיל אם ביחס הכפל והשתי שלישיות מן השלשה והשמנה | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle1:\left(2+\frac{2}{4}\right)\longrightarrow{\color{blue}{4:10}}</math> | + | :*The double superbiquartan ratio, which is not absolute - from four and ten. |
| + | ::<math>\scriptstyle1:\left(2+\frac{2}{4}\right)\longrightarrow{\color{blue}{4:10}}</math> | ||
|style="text-align:right;"|וביחס הכפל והשתי רביעיות והוא בלתי גמור בארבעה והעשרה | |style="text-align:right;"|וביחס הכפל והשתי רביעיות והוא בלתי גמור בארבעה והעשרה | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle1:\left(2+\frac{2}{5}\right)\longrightarrow{\color{blue}{5:12}}</math> | + | :*The double superbiquintan ratio - from five and twelve. |
| + | ::<math>\scriptstyle1:\left(2+\frac{2}{5}\right)\longrightarrow{\color{blue}{5:12}}</math> | ||
|style="text-align:right;"|וביחס הכפל והשתי חמישיות מן החמשה והשנים עשר | |style="text-align:right;"|וביחס הכפל והשתי חמישיות מן החמשה והשנים עשר | ||
|- | |- | ||
| | | | ||
| + | :The greater are also added two by two, and the less [are added] one by one. | ||
|style="text-align:right;"|והנה נמצא המוסיפים ג"כ יוסיפו בשנים שנים והחסרים באחד אחד | |style="text-align:right;"|והנה נמצא המוסיפים ג"כ יוסיפו בשנים שנים והחסרים באחד אחד | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|ונמצא הסדר והמנהג בשער אחד כמו סדר המספרים המונחים לשני דמיונים ושתי שלישיות | + | :The sequence and the order [of the additions] are found in one category as the sequence of the numbers given in the double [superparticular] ratio - the less and the greater are added by their number, yet the numbers of the less are as in the [double superbitertian] ratio, double superbiquartan ratio, the double superbiquintan ratio, and the rest of them. |
| + | |style="text-align:right;"|ונמצא הסדר והמנהג בשער אחד כמו סדר המספרים המונחים לשני דמיונים ושתי שלישיות ונמצא החסרים והמוסיפים יוסיפו על מספרם אלא שאנחנו נמצא מספר החסרים כמו שהיה בדמיון ושליש וכפל ושתי רביעיות וכפל ושתי חמישיות ושאר אלו‫<ref>marg. א"ב נראה שהרצון הוא כפל מה שהיה בדמיון ושליש כי לא יצדק זה בכפל ושליש במוסיפי' כי אם בחסרים</ref> | ||
| + | |- | ||
| + | | | ||
| + | *The double supertripartient | ||
| + | |style="text-align:right;"|וכאשר נרצה הכפל והשלשה חלקים | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :The first of them is the three quarters [the double supertriquartan]. |
| + | |style="text-align:right;"|והראשון שבהם שלשה רביעיות | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :The production is by the same way, except that for the three parts [the supertripartient] three are added, and for the four parts [the superquadripartient] four [are added]. |
| + | |style="text-align:right;"|הנה ההולדה על זה הדרך בעצמו אלא שאנחנו נוסיף למוסיף שלשה חלקים שלשה ולמוסיף ארבעה חלקים ארבעה | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle1:\left(2+\frac{3}{4}\right)\longrightarrow{\color{blue}{4:11}}</math> | + | :*The beginning of the double supertripartient is the double supertriquartan - starting from four and eleven. |
| + | ::<math>\scriptstyle1:\left(2+\frac{3}{4}\right)\longrightarrow{\color{blue}{4:11}}</math> | ||
|style="text-align:right;"|והנה הראשון שבכפל ושלשה חלקים הכפל ושלשה רביעיות והתחלתו מן הארבעה והאחד עשר | |style="text-align:right;"|והנה הראשון שבכפל ושלשה חלקים הכפל ושלשה רביעיות והתחלתו מן הארבעה והאחד עשר | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle1:\left(2+\frac{3}{5}\right)\longrightarrow{\color{blue}{5:13}}</math> | + | :*The double supertriquintan - starting from five and thirteen. |
| − | |style="text-align:right;"|אח"כ הכפל ושלשה חמישיות והתחלתו מן החמשה ושלשה עשר ועל הדרך הזה | + | ::<math>\scriptstyle1:\left(2+\frac{3}{5}\right)\longrightarrow{\color{blue}{5:13}}</math> |
| + | |style="text-align:right;"|אח"כ הכפל ושלשה חמישיות והתחלתו מן החמשה ושלשה עשר | ||
| + | |- | ||
| + | | | ||
| + | :So on in this way. | ||
| + | |style="text-align:right;"|ועל הדרך הזה | ||
|- | |- | ||
| | | | ||
| + | :The excesses of the terms are as before. | ||
|style="text-align:right;"|והנה נמצא תוספת מדרגות המספרים כמו שהיה | |style="text-align:right;"|והנה נמצא תוספת מדרגות המספרים כמו שהיה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|וכאשר נתנהג במה שבשער | + | :When referring to one category, the less and the greater are added as themselves also. |
| + | |style="text-align:right;"|וכאשר נתנהג במה שבשער אחד נמצא החסרים והמוסיפים ג"כ יתוספו כמו עצמם | ||
|- | |- | ||
| | | | ||
| + | :The number of the less is as it is and the number of the greater is [another?] number. | ||
|style="text-align:right;"|ואמנם מספר החסרים יהיה כמו שהיה ומספר המוסיפים מספר אחד | |style="text-align:right;"|ואמנם מספר החסרים יהיה כמו שהיה ומספר המוסיפים מספר אחד | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|וכאשר נרצה יחס שלשה כפלים וחלק או שני חלקים אחרים נעשה בהולדת אלו מה שכבר עשינו אלא שאנחנו לא נכפול פעם אחת לבד אבל במספר אותם הכפלים אח"כ נעשה בחלק והחלקים מה שכבר עשינו | + | *The triple superparticular ratios, the triple superbitertian ratios, or the triple superpartient ratios |
| + | |style="text-align:right;"|וכאשר נרצה יחס שלשה כפלים וחלק או שני חלקים אחרים | ||
| + | |- | ||
| + | | | ||
| + | :The production of these is as already done, except for not doubling once, but multiplying by the number of the multiples, then the part or the parts are treated as before. | ||
| + | |style="text-align:right;"|נעשה בהולדת אלו מה שכבר עשינו אלא שאנחנו לא נכפול פעם אחת לבד אבל במספר אותם הכפלים אח"כ נעשה בחלק והחלקים מה שכבר עשינו | ||
| + | |- | ||
| + | | | ||
| + | :*The triple superparticular ratios | ||
| + | | | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle1:\left(3+\frac{1}{3}\right)\longrightarrow{\color{blue}{3:10}}</math> | + | ::*The beginning of the triple sesquitertian is three and ten. |
| + | :::<math>\scriptstyle1:\left(3+\frac{1}{3}\right)\longrightarrow{\color{blue}{3:10}}</math> | ||
|style="text-align:right;"|ונמצא ראשית השלשה כפלים ושליש משלשה ועשרה | |style="text-align:right;"|ונמצא ראשית השלשה כפלים ושליש משלשה ועשרה | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle1:\left(3+\frac{1}{4}\right)\longrightarrow{\color{blue}{4:13}}</math> | + | ::*The beginning of the triple sesquiquartan is four and thirteen. |
| + | :::<math>\scriptstyle1:\left(3+\frac{1}{4}\right)\longrightarrow{\color{blue}{4:13}}</math> | ||
|style="text-align:right;"|וראשית שלשה כפלים ורובע מארבעה ושלשה עשר | |style="text-align:right;"|וראשית שלשה כפלים ורובע מארבעה ושלשה עשר | ||
|- | |- | ||
| | | | ||
| + | ::The less are added one by one and the greater three by three. | ||
|style="text-align:right;"|ונמצא החסרים יוסיפו באחד אחד והמוסיפים בשלשה שלשה | |style="text-align:right;"|ונמצא החסרים יוסיפו באחד אחד והמוסיפים בשלשה שלשה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|וכאשר נקח ברחב נמצא ראשית שלשה כפלים וחצי משנים ושבעה ושנית מארבעה וארבעה עשר ונמצא ג"כ כלם יתוספו במספרם | + | ::When taken breadthwise: |
| + | |style="text-align:right;"|וכאשר נקח ברחב | ||
| + | |- | ||
| + | | | ||
| + | ::*The triple sesquialter: <math>\scriptstyle{\color{blue}{2:7;\quad 4:14}}</math> | ||
| + | |style="text-align:right;"|נמצא ראשית שלשה כפלים וחצי משנים ושבעה ושנית מארבעה וארבעה עשר | ||
| + | |- | ||
| + | | | ||
| + | ::All are also added by their number. | ||
| + | |style="text-align:right;"|ונמצא ג"כ כלם יתוספו במספרם | ||
|- | |- | ||
| | | | ||
| + | ::The less follow the addition of the successive even numbers. | ||
|style="text-align:right;"|והחסר ירוץ על תוספת הזוגות הנמשכים | |style="text-align:right;"|והחסר ירוץ על תוספת הזוגות הנמשכים | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|ונמצא ראשית שלשה כפלים ושליש מהשלשה והעשרה ושנית מהששה ועשרים | + | ::*The triple sesquitertian: <math>\scriptstyle{\color{blue}{3:10;\quad 6:20}}</math> |
| + | |style="text-align:right;"|ונמצא ראשית שלשה כפלים ושליש מהשלשה והעשרה ושנית מהששה ועשרים | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::The rule is kept. |
| + | |style="text-align:right;"|ונמצא השרש שמור | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle1:\left(3+\frac{2}{4}\right)\longrightarrow{\color{blue}{4:14}}</math> | + | :*The triple superbipartient |
| + | |style="text-align:right;"|וכאשר יבחנו השלשה כפלים והשני חלקים | ||
| + | |- | ||
| + | | | ||
| + | ::*Their beginning is the triple superbitertian - starting from three and eleven. | ||
| + | :::<math>\scriptstyle1:\left(3+\frac{2}{3}\right)\longrightarrow{\color{blue}{3:11}}</math> | ||
| + | |style="text-align:right;"|יהיה ראשיתם שלשה כפלים ושני שלישיות והראשון משלשה ואחד עשר | ||
| + | |- | ||
| + | | | ||
| + | ::*The beginning of the triple superbiquartan from four and fourteen. | ||
| + | :::<math>\scriptstyle1:\left(3+\frac{2}{4}\right)\longrightarrow{\color{blue}{4:14}}</math> | ||
|style="text-align:right;"|וראשית שלשה כפלים ושני רביעיות מארבעה וארבעה עשר | |style="text-align:right;"|וראשית שלשה כפלים ושני רביעיות מארבעה וארבעה עשר | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle1:\left(3+\frac{2}{5}\right)\longrightarrow{\color{blue}{5:17}}</math> | + | ::*The beginning of the triple superbiquintan from five and seventeen. |
| + | :::<math>\scriptstyle1:\left(3+\frac{2}{5}\right)\longrightarrow{\color{blue}{5:17}}</math> | ||
|style="text-align:right;"|וראשית שלשה כפלים ושתי חמישיות מחמשה ושבעה עשר | |style="text-align:right;"|וראשית שלשה כפלים ושתי חמישיות מחמשה ושבעה עשר | ||
|- | |- | ||
| | | | ||
| + | ::The excesses of the less are by the sequence of the natural numbers and the greater [are added] three by three. | ||
|style="text-align:right;"|ונמצא ההעדף בחסרים על משך המספרים הטבעיים והמוסיפים בשלשה שלשה | |style="text-align:right;"|ונמצא ההעדף בחסרים על משך המספרים הטבעיים והמוסיפים בשלשה שלשה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|וכאשר נקח ברוחב | + | ::When taken breadthwise: |
| + | |style="text-align:right;"|וכאשר נקח ברוחב | ||
|- | |- | ||
| | | | ||
| + | ::*The triple superbitertian: <math>\scriptstyle{\color{blue}{3:11;\quad 6:22}}</math> | ||
| + | |style="text-align:right;"|נמצא ראשית שלשה כפלים ושתי שלישיות משלשה ואחד עשר ושנית מששה ושנים ועשרים | ||
| + | |- | ||
| + | | | ||
| + | ::The order is kept. | ||
| + | |style="text-align:right;"|והנה שמרו הסדר | ||
| + | |- | ||
| + | | | ||
| + | :*The triple supertripartient | ||
|style="text-align:right;"|וכאשר נבחין השלשה כפלים ושלשה חלקים | |style="text-align:right;"|וכאשר נבחין השלשה כפלים ושלשה חלקים | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle1:\left(3+\frac{3}{4}\right)\longrightarrow{\color{blue}{4:15}}</math> | + | ::*The beginning is the triple supertriquartan - starting from four and fifteen. |
| + | :::<math>\scriptstyle1:\left(3+\frac{3}{4}\right)\longrightarrow{\color{blue}{4:15}}</math> | ||
|style="text-align:right;"|היה ראשית זה שלשה כפלים ושלשה רביעיות וראשיתם מארבעה וחמשה עשר | |style="text-align:right;"|היה ראשית זה שלשה כפלים ושלשה רביעיות וראשיתם מארבעה וחמשה עשר | ||
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle1:\left(3+\frac{3}{5}\right)\longrightarrow{\color{blue}{5:18}}</math> | + | ::*The triple supertriquintan - starting from five and eighteen. |
| − | |style="text-align:right;"|אח"כ שלשה כפלים ושלשה חמישיות וראשיתם מחמשה ושמנה עשר ונמצא הענין על הדרך הזה | + | :::<math>\scriptstyle1:\left(3+\frac{3}{5}\right)\longrightarrow{\color{blue}{5:18}}</math> |
| + | |style="text-align:right;"|אח"כ שלשה כפלים ושלשה חמישיות וראשיתם מחמשה ושמנה עשר | ||
| + | |- | ||
| + | | | ||
| + | ::The property is in this way. | ||
| + | |style="text-align:right;"|ונמצא הענין על הדרך הזה | ||
| + | |- | ||
| + | | | ||
| + | ::When examined breadthwise: | ||
| + | |style="text-align:right;"|וכאשר נבחין ברוחב | ||
| + | |- | ||
| + | | | ||
| + | ::*The triple supertriquartan: <math>\scriptstyle{\color{blue}{4:15;\quad 8:30}}</math> | ||
| + | |style="text-align:right;"|נמצא ראשית שלשה ושלשה רביעיות מארבעה וחמשה עשר ושנית משמנה ושלשים | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::The order is kept. |
| + | |style="text-align:right;"|ונמצא זה הסדור שמור | ||
| + | |} | ||
| + | {| | ||
|- | |- | ||
| | | | ||
| + | |||
| + | ==== Tables of Ratios ==== | ||
| + | |||
| + | | | ||
| + | |- | ||
| + | |We should elaborate on this as well as establish the relations of the tens [?] by tens and extract them, but we will be satisfied with this and mention indications from the aspect of the tables with which these are associated. | ||
|style="text-align:right;"|ולנו שנוסיף בזה ונישב ג"כ התיחסות הכלל בכלל ונוציא אותו אבל אנחנו נסתפק על זה ונזכור רמזים על צד הלוחות ישתתפו בהם אלו | |style="text-align:right;"|ולנו שנוסיף בזה ונישב ג"כ התיחסות הכלל בכלל ונוציא אותו אבל אנחנו נסתפק על זה ונזכור רמזים על צד הלוחות ישתתפו בהם אלו | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|והנה מזה שאנחנו כאשר נעשה לוח משתי שורות אחת מהן ימשכו בה הנפרדים הנמשכים מתחילים מן החמשה ויעמדו אצל אחד ועשרים והשנית ימשכו בה מספרים מתחילים משלשה ויעמדו אצל אחד עשר ויתבאר לנו שבמה שבין אלו יחסים | + | *Setting a table of two columns |
| + | |style="text-align:right;"|והנה מזה שאנחנו כאשר נעשה לוח משתי שורות | ||
| + | |- | ||
| + | | | ||
| + | :*In one of them the successive odds, starting from five and ending at twenty-one. | ||
| + | |style="text-align:right;"|אחת מהן ימשכו בה הנפרדים הנמשכים מתחילים מן החמשה ויעמדו אצל אחד ועשרים | ||
| + | |- | ||
| + | | | ||
| + | :*In the second the successive numbers, starting from three and ending at eleven. | ||
| + | |style="text-align:right;"|והשנית ימשכו בה מספרים מתחילים משלשה ויעמדו אצל אחד עשר | ||
| + | |- | ||
| + | | | ||
| + | :From what is between them, ratios will be explained: | ||
| + | |style="text-align:right;"|ויתבאר לנו שבמה שבין אלו יחסים | ||
|- | |- | ||
| | | | ||
| − | {|class="wikitable" style="color: blue; text-align:center;" | + | :{|class="wikitable" style="color: blue; text-align:center;" |
|- | |- | ||
|3||5 | |3||5 | ||
| Line 2,414: | Line 2,944: | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|וכאשר נבחין מה שבכל בית מהלוח הראשון מצורף לבן גילו מהלוח האחר הנה יהיה על יחס הדמיון ושתי שלישיות | + | :*Observing what is in each rubric of the first column related to its corresponding in the other column: |
| + | |style="text-align:right;"|וכאשר נבחין מה שבכל בית מהלוח הראשון מצורף לבן גילו מהלוח האחר | ||
| + | |- | ||
| + | | | ||
| + | ::*It is in the superbitertian ratio | ||
| + | |style="text-align:right;"|הנה יהיה על יחס הדמיון ושתי שלישיות | ||
|- | |- | ||
| | | | ||
| + | ::*Then the supertriquartan ratio | ||
|style="text-align:right;"|אח"כ הדמיון ושלשה רביעיות | |style="text-align:right;"|אח"כ הדמיון ושלשה רביעיות | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|אח"כ הדמיון וארבעה חמישיות ועל הדרך הזה | + | ::*Then the superquadriquintan ratio |
| + | |style="text-align:right;"|אח"כ הדמיון וארבעה חמישיות | ||
| + | |- | ||
| + | | | ||
| + | ::And so on. | ||
| + | |style="text-align:right;"|ועל הדרך הזה | ||
|- | |- | ||
| | | | ||
| + | :*Observing the sequence of what is in the first column: they are in the absolute superbipartient ratio. | ||
|style="text-align:right;"|וכאשר נבחין סדור מה שבלוח הראשון יהיו על יחס הדמיון ושני חלקים הגמור‫<ref>marg. א"ב ר"ל שיחס כ"א אל י"ט וי"ט לי"ז וי"ז לט"ו הם יחסי הדמיון ושני חלקים והם גמורים וכן השאר</ref> | |style="text-align:right;"|וכאשר נבחין סדור מה שבלוח הראשון יהיו על יחס הדמיון ושני חלקים הגמור‫<ref>marg. א"ב ר"ל שיחס כ"א אל י"ט וי"ט לי"ז וי"ז לט"ו הם יחסי הדמיון ושני חלקים והם גמורים וכן השאר</ref> | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|וכאשר נבחין סדור מה שבלוח השני יהיו על יחס המוסיף חלק‫<ref>marg.א"ב ר"ל שיחסי י"א אל י' וי' אל ט' וט' אל ח' הם יחסי הדמיון המוסיף חלק וכן השאר</ref> | + | :*Observing the sequence of what is in the second column: they are in the superparticular ratio. |
| + | |style="text-align:right;"|וכאשר נבחין סדור מה שבלוח השני יהיו על {{#annot:term|1659,125|xYrQ}}יחס המוסיף חלק{{#annotend:xYrQ}}‫<ref>marg.א"ב ר"ל שיחסי י"א אל י' וי' אל ט' וט' אל ח' הם {{#annot:term|1659,125|GNgl}}יחסי הדמיון המוסיף חלק{{#annotend:GNgl}} וכן השאר</ref> | ||
|- | |- | ||
| | | | ||
| + | *When placing instead of the second [column], starting from three, another [column], starting from two and following the sequence of the natural numbers, the ratio of the first rubric in the first column to its corresponding in the second [column] is the double superparticular ratio. | ||
|style="text-align:right;"|וכאשר נניח תמורת הבית השני המתחיל מן הג' בית אחר מתחיל מן הב' וילך על משך המספרים אשר בטבע יהיה יחס הבית הראשון מהשורה הראשונה לבן גילו מן השנית על יחס שני דמיונים והחלק | |style="text-align:right;"|וכאשר נניח תמורת הבית השני המתחיל מן הג' בית אחר מתחיל מן הב' וילך על משך המספרים אשר בטבע יהיה יחס הבית הראשון מהשורה הראשונה לבן גילו מן השנית על יחס שני דמיונים והחלק | ||
|- | |- | ||
| − | | | + | |We should extract from this the tables of the other remaining ratios, since the first column is shared by all the ratios. |
| − | |style="text-align:right;"|ולנו שנוציא מזה הלוחות | + | |style="text-align:right;"|ולנו שנוציא מזה הלוחות לשאר היחסים הנשארים עם היות שהלוח הראשון<ref>marg. א"ב ר"ל הלוח הגדול הנרשם למעלה בבתי עשר על עשר</ref> ישתתף לכל היחסי‫' |
|- | |- | ||
| | | | ||
| Line 2,480: | Line 3,024: | ||
|- | |- | ||
| | | | ||
| + | *[<math>\scriptstyle{\color{blue}{C_3:C_2}}</math>]:The superparticular ratio is resulted, as already known, from the third and second columns. | ||
|style="text-align:right;"|ויצא אלינו יחס הדמיון והחלק ממה שכבר ידעת מהטור השלישי והשני | |style="text-align:right;"|ויצא אלינו יחס הדמיון והחלק ממה שכבר ידעת מהטור השלישי והשני | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|ויחס הדמיון ושני חלקים מהטור החמישי והשלישי והוא הדמיון ושתי שלישיות | + | *The superbipartient ratios: |
| + | |style="text-align:right;"|ויחס הדמיון ושני חלקים | ||
| + | |- | ||
| + | | | ||
| + | :*[<math>\scriptstyle{\color{blue}{C_5:C_3}}</math>]: The superbitertian ratio from the fifth and the third columns. | ||
| + | |style="text-align:right;"|מהטור החמישי והשלישי והוא הדמיון ושתי שלישיות | ||
|- | |- | ||
| | | | ||
| + | :*[<math>\scriptstyle{\color{blue}{C_6:C_4}}</math>]: The superbiquartan ratio from the sixth and the fourth columns. | ||
|style="text-align:right;"|ומן הטור הששי והרביעי והוא אל הדמיון ושני רביעיות | |style="text-align:right;"|ומן הטור הששי והרביעי והוא אל הדמיון ושני רביעיות | ||
|- | |- | ||
| | | | ||
| + | :*[<math>\scriptstyle{\color{blue}{C_7:C_5}}</math>]: The superbiquintan ratio from the seventh and the fifth columns. | ||
|style="text-align:right;"|ומהטור השביעי והחמישי והוא אל הדמיון ושתי חמישיות | |style="text-align:right;"|ומהטור השביעי והחמישי והוא אל הדמיון ושתי חמישיות | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|ועל הדרך הזה ויצא אלינו מן הלוח הטור השביעי והרביעי בהנחת שני טורים יחס הדמיון ושלשה רביעיות | + | :And so on. |
| + | |style="text-align:right;"|ועל הדרך הזה | ||
| + | |- | ||
| + | | | ||
| + | *[The supertripartient ratios:] | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :*[<math>\scriptstyle{\color{blue}{C_7:C_4}}</math>]: From the seventh and the fourth columns = the supertriquartan ratio. | ||
| + | |style="text-align:right;"|ויצא אלינו מן הלוח הטור השביעי והרביעי בהנחת שני טורים יחס הדמיון ושלשה רביעיות | ||
|- | |- | ||
| | | | ||
| + | :*[<math>\scriptstyle{\color{blue}{C_8:C_5}}</math>]: From the eighth and the fifth columns - the supertriquintan ratio. | ||
|style="text-align:right;"|ומן הטור השמיני והחמישי בהנחת שני טורים יחס הדמיון ושלשה חמישיות | |style="text-align:right;"|ומן הטור השמיני והחמישי בהנחת שני טורים יחס הדמיון ושלשה חמישיות | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|ועל הדרך הזה | + | :And so on. |
| + | |style="text-align:right;"|ועל הדרך הזה | ||
|- | |- | ||
| | | | ||
| + | *[The superquadripartient ratios:] | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :*[<math>\scriptstyle{\color{blue}{C_9:C_5}}</math>]: From the ninth and the fifth columns - the superquadriquintan ratio. | ||
| + | |style="text-align:right;"|ויצא אלינו מן הלוח התשיעי והחמישי בהנחת שלשה טורים יחס הדמיון וארבעה חמישיות | ||
| + | |- | ||
| + | | | ||
| + | :*[<math>\scriptstyle{\color{blue}{C_{10}:C_6}}</math>]: From the tenth and the sixth columns - the superquadrisextan ratio. | ||
|style="text-align:right;"|ומן הטור העשירי והששי יחס הדמיון וארבעה ששיות | |style="text-align:right;"|ומן הטור העשירי והששי יחס הדמיון וארבעה ששיות | ||
|- | |- | ||
| | | | ||
| + | *According to this way, the double superparticular ratios are also extracted from this table: | ||
|style="text-align:right;"|ועל הדרך הזה יצא לנו יחס השני דמיונים וחלק מזה הלוח ג"כ | |style="text-align:right;"|ועל הדרך הזה יצא לנו יחס השני דמיונים וחלק מזה הלוח ג"כ | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|אם הראשון שבהם והנה הוא יחס השני | + | :*[<math>\scriptstyle{\color{blue}{C_5:C_2}}</math>]: The first of them, the double sesquialter ratio, from the fifth and the second columns. |
| + | |style="text-align:right;"|אם הראשון שבהם והנה הוא יחס השני דמיונים והחצי בהנחת שני טורים מהטור החמישי והטור השני | ||
|- | |- | ||
| | | | ||
| + | :*[<math>\scriptstyle{\color{blue}{C_7:C_3}}</math>]: The second, the double sesquitertian ratio, from the seventh and the third columns. | ||
|style="text-align:right;"|ואם השני והוא יחס שני דמיונים ושליש הנה מהטור השביעי והשלישי כאשר תניח שלשה | |style="text-align:right;"|ואם השני והוא יחס שני דמיונים ושליש הנה מהטור השביעי והשלישי כאשר תניח שלשה | ||
|- | |- | ||
| | | | ||
| + | :*[<math>\scriptstyle{\color{blue}{C_9:C_4}}</math>]: The third, the double sesquiquartan ratio, from the ninth and the fourth columns. | ||
|style="text-align:right;"|ואם השלישי והוא יחס השני דמיונים ורביע מהטור התשיעי והרביעי כאשר תניח ארבעה | |style="text-align:right;"|ואם השלישי והוא יחס השני דמיונים ורביע מהטור התשיעי והרביעי כאשר תניח ארבעה | ||
|- | |- | ||
| | | | ||
| + | *The double superbipartient ratios: | ||
|style="text-align:right;"|ויצא לנו יחס השני דמיוני' והשני חלקים | |style="text-align:right;"|ויצא לנו יחס השני דמיוני' והשני חלקים | ||
|- | |- | ||
| | | | ||
| + | :*[<math>\scriptstyle{\color{blue}{C_8:C_3}}</math>]: The double superbitertian [ratio], from the eighth and the third [columns]. | ||
|style="text-align:right;"|אם השני שלישיות הנה מהשמיני והשלישי | |style="text-align:right;"|אם השני שלישיות הנה מהשמיני והשלישי | ||
|- | |- | ||
| | | | ||
| + | :*[<math>\scriptstyle{\color{blue}{C_{10}:C_4}}</math>]: The double superbiquartan [ratio], from the tenth and the fourth [columns]. | ||
|style="text-align:right;"|והשני רביעיו' מהעשירי והרביעי | |style="text-align:right;"|והשני רביעיו' מהעשירי והרביעי | ||
|- | |- | ||
| | | | ||
| + | *The [double] supertripartient ratios and the rest of the ratios are extracted when conducting in the way that was established. | ||
|style="text-align:right;"|ויצא לנו יחס הדמיון ושלשה חלקים ושאר היחסים כאשר נתנהג בדרך אשר כוננו אליה | |style="text-align:right;"|ויצא לנו יחס הדמיון ושלשה חלקים ושאר היחסים כאשר נתנהג בדרך אשר כוננו אליה | ||
|- | |- | ||
| | | | ||
| + | |||
| + | ==== Producing the Ratios from Equality ==== | ||
| + | |||
| + | | | ||
| + | |- | ||
| + | |The ancients referred to the method of production the ratios from equality and the extraction of the different ratios from the referred ratios. | ||
|style="text-align:right;"|וכבר רמזו הקדמוני' אל דרך התילד משווי היחסים והביא אל היחסים המתחלפים מהיחסי' הרמוז אליהם | |style="text-align:right;"|וכבר רמזו הקדמוני' אל דרך התילד משווי היחסים והביא אל היחסים המתחלפים מהיחסי' הרמוז אליהם | ||
|- | |- | ||
| − | | | + | |Which is, that whichever equal numbers, out of which three will be arranged, all ratios can be produced from them by the method employed with them: |
|style="text-align:right;"|והיא כי איזה מספרים שוים יסודרו מהם שלשה אפשר שיתילדו היחסים כלם מהם בדרך נעשה בהם | |style="text-align:right;"|והיא כי איזה מספרים שוים יסודרו מהם שלשה אפשר שיתילדו היחסים כלם מהם בדרך נעשה בהם | ||
|- | |- | ||
| | | | ||
| + | :A table with three numbers, then three other number, three by three. | ||
|style="text-align:right;"|והנה יהיה לוח בו שלשה מספרים אח"כ שלשה מספרים אחרים ויהיו שלשה שלשה | |style="text-align:right;"|והנה יהיה לוח בו שלשה מספרים אח"כ שלשה מספרים אחרים ויהיו שלשה שלשה | ||
|- | |- | ||
| | | | ||
| − | {|class="wikitable" style="color: blue; text-align:center;" | + | :They are multiplied for examination and figurative illustration for the experience. |
| + | |style="text-align:right;"|ויתרבו לבחינה וההרחבה בנסיון | ||
| + | |- | ||
| + | | | ||
| + | :It is joined breadthwise by another table divided similarly. | ||
| + | |style="text-align:right;"|וימשך אליו ברוחב לוח אחר נחלק בחלקיו | ||
| + | |- | ||
| + | | | ||
| + | :{|class="wikitable" style="color: blue; text-align:center;" | ||
|- | |- | ||
|4||2||1||rowspan="4"| ||1||1||1 | |4||2||1||rowspan="4"| ||1||1||1 | ||
| Line 2,560: | Line 3,155: | ||
|} | |} | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| + | *Taking the first and placing it in the first rubric of each line breadthwise [<math>\scriptstyle{\color{blue}{b_1=a_1}}</math>] | ||
|style="text-align:right;"|ונאמר שאנחנו כאשר נקח הראשון ונניחהו בבית מכל טור ברחב על שהוא ראשון | |style="text-align:right;"|ונאמר שאנחנו כאשר נקח הראשון ונניחהו בבית מכל טור ברחב על שהוא ראשון | ||
|- | |- | ||
| | | | ||
| + | *Summing the first and the second and placing the [sum] in the second rubric of the second table, so there is 2 in the line of the units [<math>\scriptstyle{\color{blue}{b_2=a_2+a_1=2}}</math>] | ||
|style="text-align:right;"|אח"כ נחבר הראשון והשני ונסדר אותו בבית שני מהלוח השני יהיה מטור האחדים שנים | |style="text-align:right;"|אח"כ נחבר הראשון והשני ונסדר אותו בבית שני מהלוח השני יהיה מטור האחדים שנים | ||
|- | |- | ||
| | | | ||
| + | *Summing the first, the third and double the second and placing the [sum] in the third rubric, so there is 4 in the line of the units [<math>\scriptstyle{\color{blue}{b_3=a_1+a_3+2a_2=4}}</math>] | ||
|style="text-align:right;"|אח"כ נחבר הראשון והשלישי וכפל השני ונסדר אותו בבית השלישי ממנו ויהיה מטור האחדים ארבעה | |style="text-align:right;"|אח"כ נחבר הראשון והשלישי וכפל השני ונסדר אותו בבית השלישי ממנו ויהיה מטור האחדים ארבעה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|אח"כ נשים הבית השני שרש ונחבר ממנו זה החבור ונעתיק אותו אל הבית השלישי זה ההעתק | + | *Placing the second rubric as a basis [<math>\scriptstyle{\color{blue}{c_1=b_2}}</math>] |
| + | |style="text-align:right;"|אח"כ נשים הבית השני שרש | ||
| + | |- | ||
| + | |? | ||
| + | |style="text-align:right;"|ונחבר ממנו זה החבור ונעתיק אותו אל הבית השלישי זה ההעתק | ||
|- | |- | ||
| − | | | + | |The additions of these rubrics proceed continually, so they are four lengthwise. |
|style="text-align:right;"|וילך בהתמדה התוספת הזה באלה הבתים והנה יהיו ארבעה בארך | |style="text-align:right;"|וילך בהתמדה התוספת הזה באלה הבתים והנה יהיו ארבעה בארך | ||
|- | |- | ||
| − | | | + | |If happened first that the ratio of every three numbers on one line is a continuous ratio. |
|style="text-align:right;"|קרה מזה ראשונה שיהיה יחס כל שלשה מספרים בשורה אחת יחס מתדבק | |style="text-align:right;"|קרה מזה ראשונה שיהיה יחס כל שלשה מספרים בשורה אחת יחס מתדבק | ||
|- | |- | ||
| − | | | + | |The required ratios are produced from them. |
|style="text-align:right;"|ויתילדו מהם מהיחסים הדרושים | |style="text-align:right;"|ויתילדו מהם מהיחסים הדרושים | ||
|- | |- | ||
| | | | ||
| + | *First the multiple ratios: | ||
|style="text-align:right;"|ראשונה יחסי הכפולים | |style="text-align:right;"|ראשונה יחסי הכפולים | ||
|- | |- | ||
| | | | ||
| + | :*[<math>\scriptstyle{\color{blue}{R_{2}}}</math>]: what is on the second row is in the double ratio. | ||
|style="text-align:right;"|ונמצא מה שבבית השני על יחסי השני דמיונים | |style="text-align:right;"|ונמצא מה שבבית השני על יחסי השני דמיונים | ||
|- | |- | ||
| | | | ||
| + | :*[<math>\scriptstyle{\color{blue}{R_{3}}}</math>]: what is on the third row is in the triple ratio. | ||
|style="text-align:right;"|ומה שבבית השלישי על יחסי השלשה כפלים | |style="text-align:right;"|ומה שבבית השלישי על יחסי השלשה כפלים | ||
|- | |- | ||
| | | | ||
| + | :*[<math>\scriptstyle{\color{blue}{R_{4}}}</math>]: what is on the fourth row is in the quadruple ratio. | ||
|style="text-align:right;"|ומה שבבית הרביעי על יחסי הארבעה כפלים | |style="text-align:right;"|ומה שבבית הרביעי על יחסי הארבעה כפלים | ||
|- | |- | ||
| | | | ||
| + | :It goes one endlessly. | ||
|style="text-align:right;"|וילך זה בהתמדה עד אין תכלית | |style="text-align:right;"|וילך זה בהתמדה עד אין תכלית | ||
|- | |- | ||
| | | | ||
| + | :*[<math>\scriptstyle{\color{blue}{R_3:R_2}}</math>]: the numbers that are on the third row are in the sesquialter ratio to what is on the second row. | ||
|style="text-align:right;"|וקרה שהיה מספר מה [שבבית השלישי על יחס המוסיף חצי למה] שבבית השני | |style="text-align:right;"|וקרה שהיה מספר מה [שבבית השלישי על יחס המוסיף חצי למה] שבבית השני | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|ומה שבבית הרביעי על יחס המוסיף שליש למה שבבית השלישי ועל הדרך הזה | + | :*[<math>\scriptstyle{\color{blue}{R_4:R_3}}</math>]: what is on the fourth row to what is on the third row are in the sesquitertian ratio. |
| + | |style="text-align:right;"|ומה שבבית הרביעי על יחס המוסיף שליש למה שבבית השלישי | ||
| + | |- | ||
| + | | | ||
| + | :And so on. | ||
| + | |style="text-align:right;"|ועל הדרך הזה | ||
|- | |- | ||
| | | | ||
| + | :*[<math>\scriptstyle{\color{blue}{C_2R_2:C_1R_1}}</math>]: what is in the second rubric of the second row to what is in the first rubric is in the quadruple ratio. | ||
|style="text-align:right;"|ומה שבבית השני מהשורה השנית על יחס ארבעה כפלים למה שבבית הראשון‫<ref>marg. ר"ל אם נקח הא' המדרגות החצי</ref> | |style="text-align:right;"|ומה שבבית השני מהשורה השנית על יחס ארבעה כפלים למה שבבית הראשון‫<ref>marg. ר"ל אם נקח הא' המדרגות החצי</ref> | ||
|- | |- | ||
| | | | ||
| + | :*[<math>\scriptstyle{\color{blue}{C_3R_3:C_2R_2}}</math>]: what is in the third rubric to what is in the second rubric is in the double sesquiquartan ratio. | ||
|style="text-align:right;"|ומה שבבית השלישי על יחס שני דמיונים ורביע למה שבבית השני | |style="text-align:right;"|ומה שבבית השלישי על יחס שני דמיונים ורביע למה שבבית השני | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|ומה שבבית הרביעי על יחס דמיון ושבעה תשיעיות למה שבבית השלישי | + | :*[<math>\scriptstyle{\color{blue}{C_3R_4:C_3R_3}}</math>]: what is in the fourth rubric to what is in the third rubric is in the superseptinona ratio. |
| + | |style="text-align:right;"|ומה שבבית הרביעי על יחס דמיון ושבעה תשיעיות למה שבבית השלישי | ||
|- | |- | ||
| − | | | + | |? |
| + | |style="text-align:right;"|לא יהיה זה סדור | ||
| + | |- | ||
| + | |When wanting to add to the diagram other ratios instead of the diagram of the multiple ratios: replacing the third column with the first and the first with the third, so the middle remains in its place. | ||
|style="text-align:right;"|וכאשר נרצה להוסיף לציור היחסים האחרים תמורת ציורנו ליחסי הכפלים נהפוך השורה השנית<ref>marg. ר"ל האמצעית</ref> באורך עד שיפול הראשון השלישי בראשון והראשון בשלישי וישאר האמצעי על ענינו | |style="text-align:right;"|וכאשר נרצה להוסיף לציור היחסים האחרים תמורת ציורנו ליחסי הכפלים נהפוך השורה השנית<ref>marg. ר"ל האמצעית</ref> באורך עד שיפול הראשון השלישי בראשון והראשון בשלישי וישאר האמצעי על ענינו | ||
|- | |- | ||
| | | | ||
| + | *Taking the first [after switching C_1 with C_3] and placing it first on the third [table] [<math>\scriptstyle{\color{blue}{b_1=a_1=4}}</math>] | ||
|style="text-align:right;"|וכאשר נקח לחבר החבור הנזכר מזה המקום בשנקח הראשון אח"כ נעתיקנו ראשונה בשורה השלישית ויהיה ארבעה | |style="text-align:right;"|וכאשר נקח לחבר החבור הנזכר מזה המקום בשנקח הראשון אח"כ נעתיקנו ראשונה בשורה השלישית ויהיה ארבעה | ||
|- | |- | ||
| | | | ||
| + | *Summing the first and the second and placing the [sum] in the third [table] [<math>\scriptstyle{\color{blue}{b_2=a_2+a_1=6}}</math>] | ||
|style="text-align:right;"|אח"כ נחבר הראשון והשני ונעתיק אותו אל השורה השלישית ויהיה ששה | |style="text-align:right;"|אח"כ נחבר הראשון והשני ונעתיק אותו אל השורה השלישית ויהיה ששה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|אח"כ נחבר הראשון | + | *Summing the first, which is 4, the third, which is 1, and double the second, which is 4, and placing the [sum] in the third rubric [<math>\scriptstyle{\color{blue}{a_1+a_3+2a_2=4+1+4=9}}</math>] |
| + | |style="text-align:right;"|אח"כ נחבר הראשון והוא ארבעה והשלישי והוא אחד וכפל השני והוא ארבעה ונעתיק אותו אל הבית השלישי והנה יהיה תשעה | ||
|- | |- | ||
| | | | ||
| + | :*The numbers of the line follow the sesquialter ratio, they are produced from the double ratio, and the are both named after the two. | ||
|style="text-align:right;"|וימשכו מספרי השורה על יחס המוסיף חצי וכבר התילדו מיחס הכפל והם הנקראים יחד על שם השנים | |style="text-align:right;"|וימשכו מספרי השורה על יחס המוסיף חצי וכבר התילדו מיחס הכפל והם הנקראים יחד על שם השנים | ||
|- | |- | ||
| | | | ||
| + | :*When this procedure is applied on the line of the triple ratio lengthwise the result are three numbers in the sesquitertian ratio, for both are named after the three. | ||
|style="text-align:right;"|וכאשר נעשה זה המעשה בשורה אשר ברחב אשר ליחס השלשה כפלים יצאו לך שלשה מספרים על יחס המוסיף שליש כי שניהם נקראים בשם השלשה | |style="text-align:right;"|וכאשר נעשה זה המעשה בשורה אשר ברחב אשר ליחס השלשה כפלים יצאו לך שלשה מספרים על יחס המוסיף שליש כי שניהם נקראים בשם השלשה | ||
|- | |- | ||
| | | | ||
| + | :*The same for the fourth line, for it will produce the sesquiquartan ratio. | ||
|style="text-align:right;"|וכן הענין בטור הרביעי כי הוא יוציא יחסי המוסיף רביעית | |style="text-align:right;"|וכן הענין בטור הרביעי כי הוא יוציא יחסי המוסיף רביעית | ||
|- | |- | ||
| | | | ||
| + | *When the setting of the numbers in sesquialter ratio is reversed, the double sesquialter ratio is produced. | ||
|style="text-align:right;"|וכאשר תהפך הנחת מספרי המוסיף חצי יולד לך יחס הכפל וחצי | |style="text-align:right;"|וכאשר תהפך הנחת מספרי המוסיף חצי יולד לך יחס הכפל וחצי | ||
|- | |- | ||
| | | | ||
| + | *And the double sesquitertian ratio [is produced] from the sesquitertian ratio. | ||
|style="text-align:right;"|ומהמוסיף שליש יחס הכפל ושליש | |style="text-align:right;"|ומהמוסיף שליש יחס הכפל ושליש | ||
| + | |- | ||
| + | |When reversing the numbers of the superparticular ratio and applying the known procedure, the rest of the ratios are produced, and they never cease to be produced from each other endlessly, until the production of all of them from equality is revealed. | ||
| + | |style="text-align:right;"|וכאשר תהפך מספרי המוסיף חלק ותנהג המנהג הידוע יצאו לך שאר היחסים ולא יסורו מלצאת לך קצתם מקצת אל בלתי תכלית עד שיתגלה התילדות כל אלו מיחס השווי | ||
|- | |- | ||
| | | | ||
| − | + | ||
| + | ==== Reducing the Ratios to Equality ==== | ||
| + | |||
| + | | | ||
|- | |- | ||
| − | | | + | |One can reverse it and find that all the other ratios are reduced to equality. |
|style="text-align:right;"|ובידך שתהפך ותמצא שאר היחסים כלם ישובו אל יחס השווי | |style="text-align:right;"|ובידך שתהפך ותמצא שאר היחסים כלם ישובו אל יחס השווי | ||
| + | |- | ||
| + | |The procedure: | ||
| + | *Assuming three numbers in a successive ratio. | ||
| + | |style="text-align:right;"|המשל בזה שאתה כאשר תניח מספרים ג' על יחס נמשך | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *Keeping the smaller as it is [<math>\scriptstyle b_1=a_1</math>]. |
| + | |style="text-align:right;"|ותשמור הקטן בעניינו | ||
|- | |- | ||
| | | | ||
| + | *Subtracting it from the mean and setting the remainder as a mean term [<math>\scriptstyle b_2=a_2-a_1</math>]. | ||
|style="text-align:right;"|אח"כ נגרע אותו מן האמצעי ותשים מה שנשאר גבול אמצעי | |style="text-align:right;"|אח"כ נגרע אותו מן האמצעי ותשים מה שנשאר גבול אמצעי | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|אח"כ תשליך אותו מהגדול כמו הקטן ודמיון כפל השני האמצעי ותשים מה שנשאר גבול שלישי | + | *Subtracting the smaller and double the second from the greater and setting the remainder as a third term [<math>\scriptstyle b_3=a_3-\left[2\left(a_2-a_1\right)+a_1\right]=a_3-\left(2b_2+b_1\right)</math>]. |
| + | |style="text-align:right;"|אח"כ תשליך אותו מהגדול כמו הקטן ודמיון כפל השני האמצעי ותשים מה שנשאר {{#annot:term|1580,1578|dFs4}}גבול{{#annotend:dFs4}} שלישי | ||
|- | |- | ||
| − | | | + | |One finds a continuous ratio. |
|style="text-align:right;"|תמצא יחס מתדבק | |style="text-align:right;"|תמצא יחס מתדבק | ||
|- | |- | ||
| − | | | + | |Apply this procedure on these numbers and terms and another ratio will be produced. |
|style="text-align:right;"|אחר כן תעשה באלה המספרים והגבולים זה הפעל ויצא לך יחס אחר | |style="text-align:right;"|אחר כן תעשה באלה המספרים והגבולים זה הפעל ויצא לך יחס אחר | ||
|- | |- | ||
| − | | | + | |According to this way until reaching the equality. |
|style="text-align:right;"|ועל הדרך הזה עד שיביא אותך אל יחס השווי | |style="text-align:right;"|ועל הדרך הזה עד שיביא אותך אל יחס השווי | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|דמיון זה שיהיו המספרים ראשונה על יחס שני דמיונים ושתי | + | ::For example: the numbers are first in the double superbitertian ratio, such as <math>\scriptstyle{\color{blue}{9;\;24;\;64}}</math> |
| + | |style="text-align:right;"|דמיון זה שיהיו המספרים ראשונה על יחס שני דמיונים ושתי שלישיות כמו ט' וכ"ד וס"ד | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|והנה | + | ::*<math>\scriptstyle{\color{blue}{b_1=a_1=9}}</math> |
| + | |style="text-align:right;"|והנה תשמור ט' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::*<math>\scriptstyle{\color{blue}{b_2=a_2-a_1=24-9=15}}</math> |
| + | |style="text-align:right;"|ותפיל אותם מכ"ד ותשים מה שנשאר והוא ט"ו גבול שני | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::*<math>\scriptstyle{\color{blue}{b_3=a_3-\left[2\left(a_2-a_1\right)+a_1\right]=64-\left[\left(2\sdot15\right)+9\right]=25}}</math> |
| + | |style="text-align:right;"|והנה תקח כפלו עם ט' ותפיל אותם מס"ד וישארו לך כ"ה והנה תשים אותם שלישי | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::The result are succesive numbers [<math>\scriptstyle{\color{red}{9;\;15;\;25}}</math>] in the superbitertian ratio. |
| + | |style="text-align:right;"|יצאו לך מספרים נמשכים על {{#annot:superbitertian|128|A9nH}}יחס המוסיף שני שלישים{{#annotend:A9nH}} | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Proceeding with [these numbers <math>\scriptstyle{\color{red}{9;\;15;\;25}}</math>] by this procedure, and the result are <math>\scriptstyle{\color{blue}{9;\;6;\;4}}</math>, which are successive numbers in the sesquialter ratio. |
| + | |style="text-align:right;"|אח"כ תעשה זה המעשה במה שאצלך ויצאו לך ט' וו' וד' והם מספרים נמשכים על יחס המוסיף חצי | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Proceeding with these numbers [<math>\scriptstyle{\color{red}{9;\;6;\;4}}</math>] by this procedure, and the result are <math>\scriptstyle{\color{blue}{4;\;2;\;1}}</math>, which are in the double ratio. |
| + | |style="text-align:right;"|אח"כ תעשה זה המעשה באלה המספרים ויצאו לד' ב' א' וזה על יחס הכפל | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Proceeding with this procedure, and the result are <math>\scriptstyle{\color{blue}{1;\;1;\;1}}</math>, restored to equality. |
| + | |style="text-align:right;"|אח"כ כשתעשה זה המעשה יצאו לך א' וא' ואחד וחזר אל יחס השווי | ||
| + | |- | ||
| + | |According to this property, when setting the triple ratio, the quadruple ratio and the other ratios that are not mentioned - reducing them to equality by reversing the method by which they were produced. | ||
| + | |style="text-align:right;"|ובזה הוא הענין כאשר תשים יחס הג' כפלים והד' כפלים ושאר היחסים אשר לא נזכרו התכה בהפך להשיב אותם על יחס השווי מהדרך אשר ממנו הרכבו | ||
|- | |- | ||
| | | | ||
| − | + | ||
| + | ==== Composing a Ratio from Two Ratios ==== | ||
| + | |||
| + | | | ||
|- | |- | ||
| − | | | + | |Moving on to the composition of a ratio from two ratios. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ונעתק עתה בחבור יחס במספרים משני יחסים |
|- | |- | ||
| − | | | + | |Prefacing a general introduction enough for examining the property in each ratio, which is that every particular example brought for the composition of a ratio from two ratios, the ratios are already found in this section by a certain description and this rule is possible for all numbers in these ratios. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ונקדים לזה הקדמה כוללת יספיק לנו ממנה בחינת הענין ביחס יחס והיא שכל משל חלקי נביא לחבור יחס במספרים משני יחסים הנה כבר נמצא היחסים בזה החלק על תאר מה והנה זה כלל עובר בכל מספרים יהיו על אלו היחסים |
|- | |- | ||
| | | | ||
| − | + | ::For example: let <math>\scriptstyle{\color{blue}{AB=4;\;AG=2;\;AD=3}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ויהיה א"ב על דרך משל ד' ויהיה א"ג ב' ויהיה א"ד ג' |
|- | |- | ||
| | | | ||
| − | * | + | ::*AB : AD = sesquitertian ratio |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ויהיה לא"ב אל א"ד יחס והוא יחס המוסיף שליש |
|- | |- | ||
| | | | ||
| − | * | + | ::*AD : AG = sesquialter ratio |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ויהיה לא"ד אל א"ג יחס והוא יחס המוסיף חצי |
|- | |- | ||
| | | | ||
| − | * | + | ::*AB : AG = double ratio, which undoubtedly consists of both these ratio. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ולא"ב אל א"ג יחס והוא יחס הכפל והוא מחבר בלי ספק משני אלו היחסים |
|- | |- | ||
| | | | ||
| − | * | + | *Proposition: for every sesquialter ratio, when a sesquitertian ratio is added to it, the sum is the same result [= double ratio]; and every double ratio bears the division and separation into these to ratios. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|הנה אומר שכל יחס המוסיף חצי יצטרף אליו יחס המוסיף שליש יהיה המקובץ מה שתתקבץ הנה בעינו וכל יחס הכפל הנה יסבול שיחלק לאלו השני יחסים ויפרד אליהם |
|- | |- | ||
| | | | ||
| − | + | :For, if not: | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|שאם לא |
|- | |- | ||
| | | | ||
| − | * | + | ::Let: |
| − | |style="text-align:right;"| | + | ::*HC : HZ = sesquialter ratio |
| + | |style="text-align:right;"|הנה יהיה יחס ה"ח לה"ז יחס המוסיף חצי | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::*HW : HC = sesquitertian ratio |
| + | |style="text-align:right;"|ויחס ה"ו לה"ח יחס המוסיף שליש | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::→ [supposition:] HW : HZ = double ratio |
| + | |style="text-align:right;"|והנה אומר שיחס ה"ו לה"ז יחס הכפל | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::[proof:] |
| + | ::*BD : WC = AD : HC | ||
| + | |style="text-align:right;"|כי אתה יודיע שכאשר נחלק הנה ירצה הבדלנו יחס ב"ד ו"ח אל א"ד ה"ח א' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :::by distinction: AG : HZ = GD : ZC |
| + | |style="text-align:right;"|ובהבדל יחס א"ג ה"ז אל ג"ד ז"ח א' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :::by equality: BD : DG = WC : CZ |
| + | |style="text-align:right;"|והנה בשווי יחס ב"ד ד"ג כמו יחס ו"ח ח"ז | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :::by composition: BG : DG = WZ : CZ |
| + | |style="text-align:right;"|ויהיה בהרכבה יחס כל ב"ג אל ד"ג וכל ו"ז אל ח"ז א' | ||
|- | |- | ||
| | | | ||
| − | | | + | :::DG : GA = CZ : ZH |
| − | + | |style="text-align:right;"|אמנם יחס ד"ג ג"א כמו ח"ז ז"ה | |
| − | |||
|- | |- | ||
| | | | ||
| − | + | :::by equality: BG : GA = WZ : ZH | |
| − | = | + | |style="text-align:right;"|הנה בדרך השווי יחס ב"ג ג"א כיחס ו"ז ז"ה |
| − | |||
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :::by expansion: AB : AG = HW : ZH |
| + | |style="text-align:right;"|הנה בדרך ההרחבה יחס א"ב א"ג הוא יחס ה"ו וז"ה | ||
|- | |- | ||
| − | | | + | |Also for the supposition of the compound ratio, it is as it is for this part or for whichever ratios. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וגם כן כאשר תהיה ההנחה היחס המורכבת הנה הוא כאשר היה בזה החלק וביחסים כמו שהיה |
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | *Setting whichever two numbers |
| + | |style="text-align:right;"|אח"כ נביא איזה שני מספרים שיהיו | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | :*HZ : HW = double ratio |
| + | |style="text-align:right;"|והנה יהיו ה"ז ה"ו ויהיו על יחס הכפל | ||
|- | |- | ||
| | | | ||
| − | + | ::→ [supposition:] The sesquialter ratio is between Z an C | |
| − | + | |style="text-align:right;"|הנה נאמר שיחס המוסיף חצי יפול בין ז' וח' | |
| − | |style="text-align:right;"|הנה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::[proof:] |
| + | ::*If not, it is external, such as Z : T | ||
| + | |style="text-align:right;"|שאם לא יפול מחוץ כמו ז' ט' | ||
|- | |- | ||
| | | | ||
| − | + | :::When attaching to them the other ratio, such as T : W, the primary compound ratio is restored. | |
| − | + | |style="text-align:right;"|והנה כאשר נצרף אליהם היחס האחר כמו ט"ו ישוב היחס המורכב הראשון | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | = | + | :::→ HW : HZ = HW : HC |
| − | + | |style="text-align:right;"|ויהיה אז יחס ה"ו ה"ז כמו יחס ה"ו ה"ח כפי מה שבארנו | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Hence, what is greater than HW is as HW [→ contradiction] |
| + | |style="text-align:right;"|והיה מה שהוא יותר גדול מן ה"ו כמו ה"ו | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::→ It is interior, such as C |
| + | |style="text-align:right;"|והנה א"כ יפול מבפנים כמו ח' | ||
| + | |- | ||
| + | |? | ||
| + | |style="text-align:right;"|ונאמר שיחס ה"ו ה"ח הוא היחס האחר שאם לא הנה יפול ה"ח עם ה"ב או ה"ח או עם ה"ט ויקרה הבטל הנזכר | ||
| + | |- | ||
| + | |We think that we brought a partial proof, as we have mentioned the sesquialter, the sesquitertian and the double ratio, but you should know that this is a general proof. | ||
| + | |style="text-align:right;"|והלא נחשוב שאנחנו הבאנו מופת חלקי לזכרנו החצי והשליש ויחס הכפל אבל ראוי שתדע שזה המופת כללי | ||
| + | |- | ||
| + | |We brought the example to give the meaning, else you can say that the two numbers AB and AG are two numbers having a certain ratio between them. | ||
| + | |style="text-align:right;"|ואמנם הבאנו משל לתת המובן שאם לא הנה לך שתאמר ששני מספרי א"ב א"ג שני מספרים חלקים וביניהם יחס מה | ||
| + | |- | ||
| + | |Thus, in this example two ratios AB : AD and AD : AG, were joined into whichever ratio it will be. | ||
| + | |style="text-align:right;"|וככה חוברנו בזה המשל משני יחסים א"ב א"ד וא"ד א"ג איזה יחס היה | ||
| + | |- | ||
| + | |? | ||
| + | |style="text-align:right;"|והנה אם נפל מספר ביניהם קטן מאחד מהם וגדול מאחר אח"כ במופת על הצד הכולל מזולת רמז אל שינוי היחס | ||
| + | |- | ||
| + | |This explanation is enough for endeavoring to confirm the proof of the composition of a ratio from two ratios by numbers as found in the teaching of music after this section. | ||
| + | |style="text-align:right;"|הנה זה הביאור יספיק ממנו ההשתדלות בקיום המופת על חבור יחס משני יחסים במספרים כאשר נמצא המשלים יוציאו אליו השני יחסים בלמודינו המוסיקי אחר זה האופן | ||
| + | |- | ||
| + | |But, we will endeavor in special explanations [to demonstrate] which ratios are as principals for the other ratios. | ||
| + | |style="text-align:right;"|ואבל אנחנו נשתדל בבאורים מיוחדים ליחס מה הם כמו ראשים לשאר היחסים | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *Proposition: the triple ratio [AD : AB] is composed from the double ratio and the sesquialter ratio. |
| + | |style="text-align:right;"|מזה שאנחנו נאמר שיחס הכפל ויחס המוסיף חצי יחובר מהם יחס הג' דימיונים א"ב | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::The proof: |
| + | |style="text-align:right;"|מופת זה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::*AG = 2AB |
| + | |style="text-align:right;"|שא"ג יכפל א"ב | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :::BG = AB = ½AG |
| + | |style="text-align:right;"|הנה ב"ג כמו א"ב הנה הוא חצי א"ג | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::*GD = ½AG |
| + | |style="text-align:right;"|אמנם ג"ד חצי א"ג | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :::AB = BG = GD |
| + | |style="text-align:right;"|הנה א"ב ב"ג ג"ד שוים קצתם לקצת | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::*→ AD = 3AB |
| + | |style="text-align:right;"|והנה יהיה כל א"ד ג' דמיוני א"ב | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *If GD = ⅓AG |
| + | |style="text-align:right;"|ואם יהיה ג"ד שליש א"ג | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Then AD = [2⅔]AB |
| + | |style="text-align:right;"|הנה א"ד כפל ושליש א"ב | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::[The proof:] Dividing AG into three segments on H and Z. |
| + | |style="text-align:right;"|והנה נחלק א"ג אל שלשה חלקים על ה' וז' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::*AH = GD = ⅓AG = ⅓(2AB) |
| + | |style="text-align:right;"|והנה יהיה א"ה כמו ג"ד והוא שליש א"ג אשר הוא כפל א"ב | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::→ ½AH = ⅓AB |
| + | |style="text-align:right;"|הנה חצי א"ה שליש א"ב | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::→ AD = AG + GD = 2AB + ⅓(2AB) |
| + | |style="text-align:right;"|הנה א"ד כמו כפל א"ב רצו' א"ג וכמו שלישית רצו' ג"ד | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *If AG : AB = sesquitertian ratio |
| + | |style="text-align:right;"|ואם היה יחס א"ג א"ב יחס המוסיף שליש | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Then AD : AB = double ratio |
| + | |style="text-align:right;"|הנה יחס א"ד א"ב יחס הכפל | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::[The proof:] Dividing AB into two segments on H. |
| + | |style="text-align:right;"|והנה נחלק א"ב בשני חלקים על ה' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::*AH = BG |
| + | |style="text-align:right;"|ויהיה א"ה כמו ב"ג | ||
|- | |- | ||
| | | | ||
| − | + | :::AH = HB = BG | |
| − | + | |style="text-align:right;"|ויהיו חלקי א"ה ה"ב ב"ג שוים והם ג' | |
| − | |||
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::*GD = one of the three segments of AG |
| + | |style="text-align:right;"|וג"ד כמו א' מג' חלקי א"ג | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :::the four segments are equal [AH = HB = BG = DG] |
| + | |style="text-align:right;"|הנה החלקים הד' שוים | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|כמו | + | ::→ BD = AB |
| + | |style="text-align:right;"|הנה כלל ב"ד כמו כלל א"ב | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::→ the excess of AD over AB is the same [i.e. AD = 2AB] |
| + | |style="text-align:right;"|והנה תוספת א"ד על א"ב בדמיון | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *If AG : AB = sesquitertian ratio |
| + | |style="text-align:right;"|ואם היה יחס א"ג א"ב יחס המוסיף שליש | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :And AD : AG = sesquioctavian ratio |
| + | |style="text-align:right;"|ויחס א"ד א"ג יחס המוסיף שמינית | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Then AD : AB = sesquialter ratio |
| + | |style="text-align:right;"|הנה יחס א"ד א"ב יחס המוסיף חצי | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::[The proof:] Dividing AB into thirds on Z and H. |
| + | |style="text-align:right;"|והנה נחלק א"ב לשלישיותיו על ז' וה' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :::AZ = ZH = HB = BG |
| + | |style="text-align:right;"|ויהיו חלקי א"ז ז"ה ה"ב ב"ג שוים והם ד' חלקים | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :::half of each is ⅛AG = GD |
| + | |style="text-align:right;"|וחצי כל אחד מהם הוא שמינית א"ג והוא שוה לג"ד | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :::BD = 3GD |
| + | |style="text-align:right;"|והנה יהיה ב"ד שלשה דמיוני ג"ד | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :::AB = 6GD |
| + | |style="text-align:right;"|וא"ב ששה דמיוני ג"ד | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :::→ [AB : BD] is sesquialter ratio |
| + | |style="text-align:right;"|והוא {{#annot:sesquialter|125|g2HQ}}יחס דמיון וחצי{{#annotend:g2HQ}} | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :::BD : DG = AB : BG |
| + | |style="text-align:right;"|ויחס ב"ד ד"ג הוא יחס א"ב ב"ג | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :::by conversion: BD : AB = DG : BG |
| + | |style="text-align:right;"|ובתמורה יחס ב"ד א"ב יחס ד"ג ב"ג | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :::DG = ½BG |
| + | |style="text-align:right;"|וד"ג חצי ב"ג | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :::→ BD = ½AB |
| + | |style="text-align:right;"|הנה ב"ד חצי א"ב | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::→ AD : AB = sesquialter ratio |
| + | |style="text-align:right;"|הנה א"ד א"ב יחס דמיון וחצי | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *If AG : AB = sesquiquartan ratio |
| + | |style="text-align:right;"|וכאשר היה יחס א"ג א"ב {{#annot:sesquiquartan|125|l13b}}יחס דמיון ורביע{{#annotend:l13b}} | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :And AD : AG = sesquiquintan ratio |
| + | |style="text-align:right;"|ויחס א"ד א"ג {{#annot:sesquiquintan|125|68dt}}דמיון וחומש{{#annotend:68dt}} | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Then AD : AB = sesquialter ratio |
| + | |style="text-align:right;"|הנה יחס א"ד א"ב יחד דמיון וחצי | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|ויהיה | + | ::[The proof:] AB is divided into quarters, each segment is the same as BG, so that the five segments are equal and BD = ½AB. |
| + | |style="text-align:right;"|לפי שא"ב כאשר יתחלק ברביעיות היה כל חלק כמו ב"ג והיו החלקים חמשה שוים ויהיה ב"ד כמו חצי א"ב | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *If AG : AB = sesquiquintan ratio |
| + | |style="text-align:right;"|וכאשר היה יחס א"ג א"ב {{#annot:sesquiquintan|125|Emnk}}יחס דמיון וחומש{{#annotend:Emnk}} | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :And AD : AG = sesquisextan ratio |
| + | |style="text-align:right;"|ויחס א"ד א"ג {{#annot:sesquisextan|125|H6fD}}יחס דמיון וששית{{#annotend:H6fD}} | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Then AD : AB = superbiquintan ratio |
| + | |style="text-align:right;"|הנה יחס א"ד א"ב יחס דמיון ושתי חמשיות | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::This is clear when AB is divided into five segments and what was done is applied. |
| + | |style="text-align:right;"|ויתבאר לך זה כשנחלק א"ב לה' חלקים ונעשה מה שעשינו | ||
| + | |- | ||
| + | |Therefore, it is clear that: | ||
| + | *The composed from the sesquisextan ratio [<math>\scriptstyle{\color{red}{7:6}}</math>] and the sesquiseptan ratio [<math>\scriptstyle{\color{red}{8:7}}</math>] is the sesquitertian ratio [<math>\scriptstyle{\color{red}{4:3}}</math>] | ||
| + | |style="text-align:right;"|ויתבאר לך מזה שיחס המחובר מ{{#annot:sesquisextan|125|y0WL}}דמיון וששית{{#annotend:y0WL}} ו{{#annot:sesquiseptan|125|imC5}}דמיון ושביעית{{#annotend:imC5}} הוא {{#annot:sesquitertian|125|h60a}}יחס דמיון ושליש{{#annotend:h60a}} | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *The composed from the sesquiseptan ratio [<math>\scriptstyle{\color{red}{8:7}}</math>] and the sesquioctavian ratio [<math>\scriptstyle{\color{red}{9:8}}</math>] is the superbiseptan ratio [<math>\scriptstyle{\color{red}{9:7}}</math>] |
| + | |style="text-align:right;"|והמחובר מדמיון ושביעית ודמיון והשמינית הוא יחס דמיון ושתי שביעיות | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *The composed from the sesquioctavian ratio [<math>\scriptstyle{\color{red}{9:8}}</math>] and the sesquinona ratio [<math>\scriptstyle{\color{red}{10:9}}</math>] is the sesquiquartan ratio [<math>\scriptstyle{\color{red}{5:4}}</math>] |
| + | |style="text-align:right;"|והמחובר מ{{#annot:sesquioctavian|125|jIRf}}דמיון ושמינית{{#annotend:jIRf}} ו{{#annot:sesquinona|125|krIu}}דמיון ותשיעית{{#annotend:krIu}} הוא {{#annot:sesquiquartan|125|EjrN}}יחס דמיון ורביעית{{#annotend:EjrN}} | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *The composed from the sesquinona ratio [<math>\scriptstyle{\color{red}{10:9}}</math>] and the sesquidecima ratio [<math>\scriptstyle{\color{red}{11:10}}</math>] is the superbinona ratio [<math>\scriptstyle{\color{red}{11:9}}</math>] |
| + | |style="text-align:right;"|והמחובר מ{{#annot:sesquinona|125|iH9T}}יחס דמיון ותשיעית{{#annotend:iH9T}} ו{{#annot:sesquidecima|125|QjiD}}דמיון ועשירית{{#annotend:QjiD}} הוא יחס דמיון ושתי תשיעיות | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *The composed from the sesquidecima ratio [<math>\scriptstyle{\color{red}{11:10}}</math>] and the sesquiundecima ratio [<math>\scriptstyle{\color{red}{12:11}}</math>] is the sesquiquintan ratio [<math>\scriptstyle{\color{red}{6:5}}</math>] |
| + | |style="text-align:right;"|והמחובר מדמיון ועשירית ודמיון וחלק מי"א הוא יחס דמיון וחומש | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *The composed from the sesquiundecima ratio [<math>\scriptstyle{\color{red}{12:11}}</math>] and the sesquiduodecima ratio [<math>\scriptstyle{\color{red}{13:12}}</math>] is the superbiundecima ratio [<math>\scriptstyle{\color{red}{13:11}}</math>] |
| + | |style="text-align:right;"|והמחובר מדמיון וחלק מי"א ודמיון וחלק מי"ב הוא יחס שני חלקים מי"א | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *The composed from the sesquiduodecima ratio [<math>\scriptstyle{\color{red}{13:12}}</math>] and the sesquitercdecima ratio [<math>\scriptstyle{\color{red}{14:13}}</math>] is the sesquisextan ratio [<math>\scriptstyle{\color{red}{7:6}}</math>] |
| + | |style="text-align:right;"|והמחובר מדמיון וחלק מי"ב ודמיון וחלק מי"ג הוא יחס הששית | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *The composed from the sesquitertdecima ratio [<math>\scriptstyle{\color{red}{14:13}}</math>] and the sesquiquartdecima ratio [<math>\scriptstyle{\color{red}{15:14}}</math>] is the superbitertdecima ratio [<math>\scriptstyle{\color{red}{15:13}}</math>] |
| + | |style="text-align:right;"|והמחובר מדמיון וחלק מי"ג ודמיון וחלק מי"ד הוא יחס משני חלקים מי"ג | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *The composed from the sesquiquartdecima ratio [<math>\scriptstyle{\color{red}{15:14}}</math>] and the sesquiquintdecima ratio [<math>\scriptstyle{\color{red}{16:15}}</math>] is the sesquiseptian ratio [<math>\scriptstyle{\color{red}{8:7}}</math>] |
| + | |style="text-align:right;"|והמחובר מיחס הדמיון וחלק מי"ד ודמיון וחלק מט"ו הוא {{#annot:sesquiseptan|125|zrEv}}יחס דמיון ושביעית{{#annotend:zrEv}} | ||
| + | |- | ||
| + | |And so on continually. | ||
| + | |style="text-align:right;"|ועל הדרך הזה כל ההמשך | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *When AG : AB = sesquidecimoquinta ratio |
| + | |style="text-align:right;"|וכאשר היו א"ג א"ב על יחס דמיון וחלק מט"ו | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :And AD : AG = sesquiquartan ratio |
| + | |style="text-align:right;"|וא"ד א"ג על {{#annot:sesquiquartan|125|ffTa}}יחס המוסיף רביעית{{#annotend:ffTa}} | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Then AD : AB = sesquitertian ratio |
| + | |style="text-align:right;"|הנה יחס א"ד א"ב דמיון ושליש | ||
|- | |- | ||
| | | | ||
| − | + | ::[The proof:] when AB is divided into 15 segments. | |
| − | + | |style="text-align:right;"|וזה לפי שאתה כאשר תחלק א"ב בט"ו חלקים | |
| − | |||
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :::AD = 16 segments. |
| + | |style="text-align:right;"|יהיה כל א"ג י"ו חלקים | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :::GD = ¼AD = 4 segments. |
| + | |style="text-align:right;"|וג"ד רביעית זה הנה הוא ד' חלקים | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :::BD = 5 segments. |
| + | |style="text-align:right;"|הנה כל ב"ד ה' חלקים | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :::AB = [15] segments. |
| + | |style="text-align:right;"|וא"ב [ט]"ו חלקים | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :::AD = 20 segments. |
| + | |style="text-align:right;"|וכל א"ד כ' חלקים | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::→ BD = ⅓AB |
| + | |style="text-align:right;"|הנה ב"ד שליש א"ב | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :The same practice when AG : AB = sesquialter ratio |
| + | |style="text-align:right;"|וכמו זאת ההנהגה הנה הוא כאשר היה א"ג א"ב על יחס המוסיף חצי | ||
| + | |- | ||
| + | |When following this way it is possible to bring a proof for the rest of the composition in music, as the previous explanation is enough for the endeavoring in all this. | ||
| + | |style="text-align:right;"|ואפשר לך כאשר תלך בזה הדרך שתביא מופת על שאר מה שבמוסיקי מהחבור לפי שהביאור הקודם יספיק לך השתדלות בזה כלו | ||
|- | |- | ||
| − | | | + | |The third section is complete. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|נשלם המאמר השלישי' |
|- | |- | ||
| | | | ||
| − | | | + | |} |
| + | |||
| + | {| | ||
|- | |- | ||
| | | | ||
| − | + | ||
| − | + | === Numbers as Geometric Shapes === | |
| | | | ||
| − | |||
|- | |- | ||
| − | | | + | |The properties of the number from the aspect of its quantity by itself were already referred to. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כבר רמזנו לך אל ענייני המספר מצד כמותו בעצמו |
|- | |- | ||
| − | | | + | |[Now] the properties of the number from the aspect of its having a quality of combination from units, by which it resembles to the measured shapes, will be referred to. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ורמזנו לך אל עניינים מענייני המספר מצד שיש לו איכות חבור מהאחדיות ידמה בו לתמונות השעוריות |
|- | |- | ||
| − | | | + | |The images of the number resemble by combinations to the measures. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כבר ידמו תמונות המספר בחבורים לשעורים |
|- | |- | ||
| − | | | + | |[They are called]: |
| − | |style="text-align:right;"| | + | *Linear Numbers |
| + | |style="text-align:right;"|ויאמר {{#annot:term|2101,2462|yUB9}}מספרים קוויים{{#annotend:yUB9}} | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *Plane Numbers |
| + | |style="text-align:right;"|ו{{#annot:term|1880,83|xBlW}}מספרים שטחיים{{#annotend:xBlW}} ו{{#annot:term|1569,83|ni0k}}מושטחים{{#annotend:ni0k}} | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *Solid Numbers |
| + | |style="text-align:right;"|ו{{#annot:term|1852,89|R25D}}מספרים גשמיים{{#annotend:R25D}} ו{{#annot:term|1851,89|lTlq}}מוגשמים{{#annotend:lTlq}} | ||
|- | |- | ||
| | | | ||
| − | + | ==== Linear Numbers ==== | |
| − | |||
| | | | ||
| − | |||
|- | |- | ||
| − | | | + | |The linear numbers begin from one and follow their natural course. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|הנה המספרים הקוויים מתחילים הם מהאחד וילכו כפי מנהגם |
|- | |- | ||
| − | | | + | |The first linear number is two, then three and so on. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ו{{#annot:term|2101,2462|C4jI}}המספר הקוי{{#annotend:C4jI}} הראשון הוא השנים אח"כ הג' ועל הדרך הזה |
|- | |- | ||
| | | | ||
| − | + | ==== Plane Numbers ==== | |
| − | |||
| | | | ||
| − | |||
|- | |- | ||
| − | | | + | |The plane [numbers] are those that can be combined to one another by a combination that resembles to the one that have a geometrical shape. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואולם המושטחי' הנה הם אשר אפשר שיחובר קצתם אל קצת חבור ידמה קצת השטים הבעל תמונה |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והמוגשמים |
|- | |- | ||
| | | | ||
| − | + | ===== Triangular Numbers ===== | |
| − | |||
| | | | ||
| − | |||
|- | |- | ||
| − | | | + | |The first plane [numbers] are the triangular numbers. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והמושטחים ראשונים הם המספרים המשלשים |
|- | |- | ||
| − | | | + | |They are the numbers that when their units are arranged in a certain arrangement they resemble to a geometrical shape that is encompassed by three sides. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והם המספרים אשר כשיסודרו אחדיהם {{#annot:term|1402,2493|frV5}}סדור{{#annotend:frV5}} מה ידמו תמונה יקיפו בה שלש צלעות |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*The first of them is three, and its shape is: |
| + | |style="text-align:right;"|והראשון שבהם שלשה וצורתם כזה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*Then six |
| + | |style="text-align:right;"|אח"כ הששה | ||
|- | |- | ||
| − | | | + | |Their shape is generated from joining a numerical line that exceeds by one over the numerical line [of the preceding triangle] |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וצורתם תתחדש מצרוף {{#annot:term|2098,2462|4qvk}}קו מספריי{{#annotend:4qvk}} מוסיף באחד על הקו המספריי אשר הוא כמו שראית |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Two is joined to one, and the first triangle is produced, which is three, so the shape is: |
| + | |style="text-align:right;"|שנים נצטרף אל האחד ונתילד המשלש הראשון והנה יהיה שלשה ותהיה הצורה בזה | ||
|- | |- | ||
| − | | | + | |According to this way, whenever a numerical line is joined, by the sequence of the numbers, a greater triangle will generated. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ועל הדרך הזה כל מה שתצטרף אל זה קו מספריי על סדר המספרים הנמשכים יתחדש משלש יותר גדול |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::For example, when joining to this a numerical line of four units, the shape will be another triangle, like this: |
| + | |style="text-align:right;"|המשל בזה כשתצרף אל זה קו מספריי מארבעה אחדויות יהיה תמונה משלש אחר כזה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*The first of the triangular numbers is 3 and its side is 2. |
| + | |style="text-align:right;"|והנה הראשון שבמשלשים ג' וצלעו ב‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*The second triangular number is 6 and its side is 3. |
| + | |style="text-align:right;"|והמשלש השני ששה וצלעו ג‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*The third triangular number is 10 and its side is 4. |
| + | |style="text-align:right;"|והמשלש השלישי י' וצלעו ד‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*The fourth triangular number is 15 and its side is 5. |
| + | |style="text-align:right;"|והמשלש הרביעי ט"ו וצלעו ה‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *Every triangle exceeds its preceding by its own side. |
| + | |style="text-align:right;"|והנה כל משלש יוסיף על אשר ימשך תחתיו כמו צלע עצמו | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *Their sides differ according to the sequence of the numbers, from one, including one, and whichever number is summed from them is a triangle [<math>\scriptstyle{\color{red}{\sum_{k=1}^n k}}</math>]. |
| + | |style="text-align:right;"|ויתחלפו צלעותיהם על סדר המספרים הנמשכים מן האחד עם הא' ואי זה מספר שיתקבץ מזה הנה הוא משלש | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *For every triangle, its side exceeds its rank by one. |
| + | |style="text-align:right;"|וכל משלש הנה צלעו יוסיף על מדרגתו בא‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::When you are told: what is the side of the tenth triangle from the beginning of the triangular numbers? |
| + | |style="text-align:right;"|וכאשר יאמר לך מה הוא צלע המשלש העשירי מראשית המספרים המשלשים | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Hence, the number of the side and the number of the rank will be one and the same [when one is included]. |
| + | |style="text-align:right;"|יהיה מספר הצלע ומספר המדרגה אחד | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *If you are told to say that it is a square, or a cube in actu, it is not a triangle, nor a pentagon, and none of those, not in potential and not in actu, only by similarity of the name - do not pay attention to what they say. |
| + | |style="text-align:right;"|ואם יאמר אליך שתאמר שהוא מרבע או מעקב בכח הנה אינו משלש ולא מחומש ולא דבר מאלו לא בכח ולא בפועל אלא בשתוף השם ולא תשגיח למה שיאמרו | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *Every triangular number is half the product of its position by the exceeding over it by one [<math>\scriptstyle{\color{red}{\sum_{k=1}^n k=\frac{1}{2}\sdot\left[n\sdot\left(n+1\right)\right]}}</math>] |
| + | |style="text-align:right;"|וכל משלש הנה הוא חצי הכאת מדרגתו במוסיף ממנו באחד | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::So, if you are told: what is the value of the fifth potential triangle? |
| + | ::<math>\scriptstyle{\color{blue}{\frac{1}{2}\sdot\left(5\sdot6\right)=\frac{1}{2}\sdot30=15}}</math> is the fifth triangle [in potential]. | ||
| + | |style="text-align:right;"|עד שאם יאמר לך מהו מספר המשלש החמשי בכח הנה תקח חמשה ותכה אותם במוסיף ממנו באחד ויהיו שלשים ותקח חציים והוא חמשה עשר והוא המשלש החמישי‫<ref>marg. א"ב ר"ל בכח כי מספר החמשה עשר שהוא מספר המשלש החמישי אינו משלש בפעל עד שיסודרו סדור ידמה לתמונה המשלשת וישים התושבת ה' ועליהם ד' ועל הד' ג' ועל הג' ב' ועל הב' א'</ref> | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *The side of a triangle is the smaller among two successive numbers, such that the product of one of them by the other is double its triangle [<math>\scriptstyle{\color{red}{n\sdot\left(n+1\right)=2\sum_{k=1}^n k}}</math>]. |
| + | |style="text-align:right;"|וכל צלע משלש הנה הוא היותר קטן שבשני מספרים נמשכים יוכה אחד מהם באחר ויהיה ממנו כפל משלשו | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::So, if you are told: what is the side of the triangle 15? |
| + | |style="text-align:right;"|עד שאם יאמר לך מה הוא צלע חמשה עשר מהמשלשים | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{2\sdot15=30=5\sdot6}}</math> → its side is five. |
| + | |style="text-align:right;"|הנה אנחנו נכפול אותו ויהיה שלשים ונבקש שני מספרים נמשכים אשר מושטחם שלשים ונמצא שהם חמשה וששה ונאמר שצלעו חמשה | ||
| + | |} | ||
| + | {| | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|ואחר | + | |
| + | ===== Square Numbers ===== | ||
| + | | | ||
| + | |- | ||
| + | |After the triangular numbers are the square numbers. | ||
| + | |style="text-align:right;"|ואחר המספרים המשלשים המספרים המרבעים | ||
| + | |- | ||
| + | |They are as already known. | ||
| + | |style="text-align:right;"|והם אשר כבר ידעת אותם | ||
| + | |- | ||
| + | |They are generated from the numerical lines, the value of each of which is equal to the number of units in it. | ||
| + | |style="text-align:right;"|והנה הם יתחדשו מ{{#annot:term|2098,2462|ZSDE}}קוים מספריים{{#annotend:ZSDE}} שוים מספרם מספר מה שבאחד מהם מן האחדים | ||
| + | |- | ||
| + | |Their sides are according to the order of the numbers, beginning from one. | ||
| + | |style="text-align:right;"|וצלעותיהם על סדר המספרים מתחילים מן האחד | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Such as: |
| + | ::*<math>\scriptstyle{\color{blue}{1=1^2}}</math> | ||
| + | |style="text-align:right;"|כמו האחד שהוא מרבע האחד | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::*<math>\scriptstyle{\color{blue}{4=2^2}}</math> |
| + | |style="text-align:right;"|והארבעה שהוא מרבע השנים | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::*<math>\scriptstyle{\color{blue}{9=3^2}}</math> |
| + | |style="text-align:right;"|והתשעה שהוא מרבע השלשה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::*<math>\scriptstyle{\color{blue}{16=4^2}}</math> |
| + | |style="text-align:right;"|והששה עשר שהוא מרבע הארבעה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::As these shapes: |
| + | |style="text-align:right;"|כפי הצורות האלו | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *Their production is from the sum of the successive odd numbers with the one. |
| + | |style="text-align:right;"|והתילדותם מקבוץ הנפרדים הנמשכים עם האחד | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Such as: |
| + | ::*<math>\scriptstyle{\color{blue}{3+1=4}}</math> which is the first of the square numbers. | ||
| + | |style="text-align:right;"|כמו השלשה והאחד שהוא ארבעה והוא ראשון המספרים המרבעים‫<ref>marg. א"ב ר"ל לפי שהאחד אינו מספר עם היותו מרבע כאשר נזכר למעלה אבל לפי שהאחד הוא הנפרד הראשון יצדק כמו כן אמרנו שמרבע האחד יתילד מדבקותו אל עצמו ואם לא יפול בזה מלת קבוץ</ref> | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::*<math>\scriptstyle{\color{blue}{3+1+5=9}}</math> which is the second square number. |
| + | |style="text-align:right;"|אח"כ האחד והשלשה והחמשה והוא תשעה והוא המספר המרבע השני | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::*<math>\scriptstyle{\color{blue}{3+1+5+7=16}}</math> which is the third square number. |
| + | |style="text-align:right;"|אח"כ האחד והשלשה והחמשה והשבעה והוא ששה עשר והוא המספר המרבע השלישי | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *Among the properties of the squares: when they are summed from the first square, their sum is greater than the last square by the sum of the preceding squares <math>\scriptstyle\sum_{k=1}^n k^2-n^2=\sum_{k=1}^{n-1} k^2</math>. |
| + | |style="text-align:right;"|ומסגלות המרבעים שאתה כאשר תקבץ אותם ממרבע האחד יהיה מקובצם יותר ממרבע האחרון כמו מה שלפניו מן המרבעים | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::*The sum of the square of one with the square of two <math>\scriptstyle{\color{blue}{1^2+2^2}}</math> exceeds the square of two <math>\scriptstyle{\color{blue}{2^2}}</math> by the square of one <math>\scriptstyle{\color{blue}{1^2}}</math>. |
| + | |style="text-align:right;"|המשל בזה שמקבץ מרבע האחד והשנים יוסיף על מרבע השנים כמו מרבע האחד | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::*The sum of the squares of one, two and three <math>\scriptstyle{\color{blue}{1^2+2^2+3^2}}</math> exceeds the square of three <math>\scriptstyle{\color{blue}{3^2}}</math> by the sum of the two squares of one and two <math>\scriptstyle{\color{blue}{1^2+2^2}}</math>. |
| + | |style="text-align:right;"|ומקובץ מרבעי האחד והשנים והשלשה יוסיף על מרבע השלשה כמו מקבץ שני מרבעי האחד והשנים | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *When the way to produce the squares was applied, it was called ''run and return'' [Ezekiel 1, 14] |
| + | |style="text-align:right;"|וכבר הוציאו להולדת המרבעים דרך יקראוהו ''רצוא ושוב''‫<ref>יחזקאל א, יד</ref> | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *That is by starting from one and summing the ranks as much as one wishes, then summing in descending [order], and the total sum is a square <math>\scriptstyle\sum_{k=1}^n k+\sum_{k=n-1}^{1} k</math>. |
| + | |style="text-align:right;"|והוא שאתה כאשר תתחיל מן האחד ותקבץ מה שתרצה מהמדרגות אח"כ תהיה נוטה יורד ומקבץ הנה מה שיהיה מקובץ זה ההוא מרבע | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::*<math>\scriptstyle{\color{blue}{1+2+1=3+1=4}}</math> which is the first square number. |
| + | |style="text-align:right;"|המשל בזה שיעלה מן האחד אל השנים ויהיו שלשה אח"כ נשוב אל האחד ויהיו ארבעה והוא מרבע ראשון | ||
|- | |- | ||
| | | | ||
| − | + | ::*<math>\scriptstyle{\color{blue}{1+2+3+2+1=9}}</math> which is the second square number. | |
| − | + | |style="text-align:right;"|אחר כן כאשר תקבץ האחד והשנים והשלשה ותצרף אליו השנים והאחד יהיו תשעה והוא מרבע שני | |
| − | |||
| − | |||
| − | | | ||
|- | |- | ||
| − | | | + | | |
| + | ::*<math>\scriptstyle{\color{blue}{1+2+3+4+3+2+1=16}}</math> which is the third square of the square numbers. | ||
| + | |style="text-align:right;"|וכאשר תעלה מן האחד והשנים והשלשה והארבעה מקובץ אח"כ תרד ותקבץ השלשה והשנים והאחד יהיה מקובץ זה ששה עשר והוא המרבע השלישי מן המרבעים המספריים | ||
|- | |- | ||
| − | | | + | | |
| + | *The completion of this way is that the sum of all the successive numbers plus the sum that is lacking the last rank is a square <math>\scriptstyle\sum_{k=1}^n k+\sum_{k=1}^{n-1} k</math>. | ||
| + | |style="text-align:right;"|ושלמות זה הדרך שמקובץ כל המספרים הנמשכים עם מקובץ מה שהוא חסר מהם כמו המדרגה האחרונה הנה הוא מרבע | ||
|- | |- | ||
| − | | | + | | |
| + | *Also, double the sum of all successive numbers minus the last number is a square <math>\scriptstyle2\sdot\left(\sum_{k=1}^n k\right)-n</math>. | ||
| + | |style="text-align:right;"|וגם כן כפל מקובץ כל מספרים נמשכים זולת המספר האחרון הנה הוא מרבע | ||
|- | |- | ||
| − | | | + | | |
| + | *[The sum of] every two successive triangles that are summed together, [beginning] from one, three, and six, is a square, and this is also the production of the squares. | ||
| + | |style="text-align:right;"|וכל שני משלשים נמשכים יתקבצו מן האחד והשלשה והששה הנה הוא מרבע וזה גם כן התילדות המרבעים | ||
|- | |- | ||
| − | | | + | | |
| + | *Every square is [generated] from a triangle of its rank plus a triangle of its rank minus one. | ||
| + | |style="text-align:right;"|ויהיה כל מרבע ממשלש במדרגתו ומשלש חסר ממדרגתו באחד | ||
|- | |- | ||
| − | | | + | | |
| + | *For every two squares, the side of one of which is multiplied by [the side of] the other, then [the product] is doubled and added to the two squares, the total sum is a square <math>\scriptstyle n^2+m^2+\left[2\sdot\left(n\sdot m\right)\right]</math>. | ||
| + | |style="text-align:right;"|וכל שני מרבעים יוכה צלע אחד מהם באחר ויכפל ויקובץ אל השני מרבעים הנה הכל מרבע‫<ref>marg. א"ב מופת זה המשפט מד' ממאמר שני לאקלידס</ref> | ||
|- | |- | ||
| − | | | + | | |
| + | ::such as: <math>\scriptstyle{\color{blue}{4+9+\left[2\sdot\left(2\sdot3\right)\right]=25}}</math> | ||
| + | |style="text-align:right;"|כמו הכאת שנים בשלשה כאשר יקובץ כפלו עם ארבעה ותשעה אשר הוא חמשה ועשרים | ||
|- | |- | ||
| − | | | + | | |
| + | *For every square, when its root is added to it twice plus one <math>\scriptstyle n^2+2n+1</math>, or when it is added to its same and a quarter of its same <math>\scriptstyle n^2+n^2+\frac{1}{4}n^2</math>, or thrice its same <math>\scriptstyle n^2+3n^2</math>, or when its three quarters are subtracted from it <math>\scriptstyle n^2-\frac{3}{4}n^2</math> - the results are squares. | ||
| + | |style="text-align:right;"|וכל מרבע יתוסף עליו גדרו פעמים ואחד או יצורף אל דמיונו ודמיון רביעיתו או שלשה דמיוניו או יחוסר ממנו שלשה רביעיותיו הנה מה שיגיע מרבע‫<ref>marg. א"ב כמו כפל ב' וא' עם ד' ויגיע ט' או כמו י"ו יצורף לדמיונו י"ו ודמיון רביעיתו ד' ויגיע ל"ו או יצורף אל ד' שלשה דמיוניו והוא י"ב ויגיע י"ו או יחוסר מי"ו שלשה רביעיו שההוא י"ב ויגיע ד'</ref> | ||
|- | |- | ||
| − | | | + | | |
| − | + | *There is no square whose half <math>\scriptstyle\frac{1}{2}n^2</math> or double <math>\scriptstyle2n^2</math> are squares. | |
| − | + | |style="text-align:right;"|ואין מרבע שיהיה חציו או כפלו מרבע | |
| − | |||
|- | |- | ||
| − | | | + | | |
| + | *The sum of the successive squares, beginning from one, is not a square at all. | ||
| + | |style="text-align:right;"|ולא יתקבצו מהמרבעים הנמשכים המתחילים מן האחד מרבע כלל | ||
|- | |- | ||
| − | | | + | |? |
| + | |style="text-align:right;"|ואין מרבע שיהיה לו שליש מן השלמים | ||
|- | |- | ||
| − | | | + | | |
| + | *The units of the number that has a root are necessarily either 1, or 4, or 5, or 6, or 9: | ||
| + | |style="text-align:right;"|ודע שאחדי המספר הנגדר לא ימלטו אם שיהיו אחד או ארבעה או חמשה או ששה או תשעה | ||
|- | |- | ||
| − | | | + | | |
| + | :*If it is 1: the units of its side are 1 or 9. | ||
| + | |style="text-align:right;"|ואם היה אחד הנה אחדי צלעו אם אחד ואם תשעה | ||
|- | |- | ||
| − | | | + | | |
| + | :*If they are 4: [the units of its side] are 2 or 8. | ||
| + | |style="text-align:right;"|ואם היה ארבעה הנה הם שנים או שמנה | ||
|- | |- | ||
| − | | | + | | |
| + | :*If they are 5: [the units of its side] are 5. | ||
| + | |style="text-align:right;"|ואם היה חמשה הנה הם חמשה | ||
|- | |- | ||
| − | | | + | | |
| − | + | :*If they are 6: [the units of its side] are 4 or 6. | |
| − | + | |style="text-align:right;"|ואם היה ששה הנה הם ארבעה או ששה | |
| − | |||
| − | |||
| − | |- | ||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*If they are 9: [the units of its side] are 3 or 7. |
| + | |style="text-align:right;"|ואם היה תשעה הנה הם שלשה או שבעה‫<ref>marg. א"ב סבת זה כי לא יצאו אחדים באחרית כי אם מהכאת אחדי' באחדים כי מהכאת עשרות או מאות ושאר המדרגות לא יצאו אחדים וקרה שמהכאת האחדים עד ט' בעצמם היו האחדים הנשארים א' ד' ה' ו' ט' והנה תמצא שמהכאת האמצעי אשר במדרגת האחדי' שהוא ה' בעצמו נשאר ה' אח"כ הנשאר מהפאות הרחוקות מהאמצעי מרחק שוה הנה ישוו אחדי גדריהם כי אחדי פ"א שהוא מרבע ט' ישוה לאחד שהוא אחדי גדר א' וט' וא' רחוקים בשוה מן החמשה וכן אחדי ס"ד וד' למספרי ח' וב' ואחדי מ"ט וט' למספרי ז' וג' ואחדי ל"ו וי"ו למספרי ו' וד'</ref> | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *The check [= modulo] of the squares, according to the way of the people of India, is necessarily either one, or four, or seven, or nine. |
| + | |style="text-align:right;"|ובחינת‫<ref>marg. א"ב צ"ע בבחינה זו פי' במאזניהם</ref> המרבעים בדרך אנשי הודו הנה לא ימלט אם שיהיה אחד או ארבעה או שבעה או תשעה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*One: either one, or eight. |
| + | |style="text-align:right;"|והנה לאחד אחד או שמנה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*Four: either two, or seven. |
| + | |style="text-align:right;"|ולארבעה שנים או שבעה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*Seven: either four, or five. |
| + | |style="text-align:right;"|ולשבעה ארבעה או חמשה | ||
|- | |- | ||
| | | | ||
| − | + | :*Nine: either three, or six, or nine. | |
| − | + | |style="text-align:right;"|ולתשעה שלשה או ששה או תשעה | |
| − | |||
| − | |style="text-align:right;"| | ||
|} | |} | ||
{| | {| | ||
| Line 3,243: | Line 4,058: | ||
| | | | ||
| − | ==== | + | ===== Polygonal Numbers ===== |
| − | + | | | |
| − | |style="text-align:right;"| | + | |- |
| + | |Following the square numbers are the pentagonal numbers | ||
| + | |style="text-align:right;"|<big>וימשכו</big> למספרים המרבעים {{#annot:term|1672,87|HHGc}}המספרים המחמשים{{#annotend:HHGc}} | ||
|- | |- | ||
| | | | ||
| − | + | *[<math>\scriptstyle{\color{blue}{5}}</math>]: The first of them is the five, which is composed as this: | |
| − | + | |style="text-align:right;"|והראשון שבהם החמשה ויתחברו כזה | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :It is the first pentagonal and its side is two. |
| + | |style="text-align:right;"|והוא ה{{#annot:term|1672,87|r6tO}}מחמש{{#annotend:r6tO}} הראשון וצלעו שנים | ||
|- | |- | ||
| | | | ||
| − | + | *[<math>\scriptstyle{\color{blue}{12}}</math>]: The second pentagonal is that whose side is the second number, which is three, so the pentagonal formed from it is twelve, like this: | |
| − | + | |style="text-align:right;"|והמחמש השני הוא אשר צלעו המספר השני והוא שלשה ויהיה המחמש המתקבץ ממנו שנים עשר כזה | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *[<math>\scriptstyle{\color{blue}{22}}</math>]: The third number, which is four, the pentagonal that is formed from it is twenty two, like this: |
| + | |style="text-align:right;"|והמספר השלישי והוא ארבעה הנה המחומש המתקבץ ממנו הוא שנים ועשרים כזה | ||
| + | |- | ||
| + | |Likewise the fourth, the fifth, the sixth, and the seventh - the sequence of their sides is as the sequence of the successive numbers. | ||
| + | |style="text-align:right;"|והרביעי והחמשי והששי והשביעי סדור צלעותיהם על {{#annot:term|1402,2576|fbLn}}סדור המספרים הנמשכים{{#annotend:fbLn}} | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *Their production is by summing the numbers exceeding three by three, beginning from one. |
| + | |style="text-align:right;"|והתילדותם מקבוץ המספרים העודפים בשלשה שלשה מתחילים מן האחד | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Such as the numbers: <math>\scriptstyle{\color{blue}{1;\;4;\;7;\;10;\;13;\;16;\;19;\;22}}</math> with the one |
| + | |style="text-align:right;"|כמו מספרי א' ד' ז' י' י"ג י"ו י"ט כ"ב עם האחד | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*<math>\scriptstyle{\color{blue}{1+4=5}}</math> which is the first pentagonal number. |
| + | |style="text-align:right;"|כי האחד עם הארבעה חמשה והוא המחמש הראשון | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*<math>\scriptstyle{\color{blue}{1+4+7=12}}</math> which is the second pentagonal number. |
| + | |style="text-align:right;"|והאחד עם הארבעה והשבעה שנים עשר והוא המחמש השני | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*<math>\scriptstyle{\color{blue}{1+4+7+10=22}}</math> which is the third pentagonal number. |
| + | |style="text-align:right;"|והאחד עם הארבעה והשבעה והעשרה שנים ועשרים והנה זה המחמש השלישי | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *They are produced by summing the squares with the triangles, meaning summing the square that is in its rank with the triangle that is in the preceding rank. |
| + | |style="text-align:right;"|וכבר יולדו מקבוץ המרבעים עם המשלשים רצוני קבוץ המרבע אשר במדרגתו עם המשלש אשר הוא למטה ממדרגתו | ||
|- | |- | ||
| | | | ||
| − | + | :*As finding that from the sum of the second square, which is nine, with the first triangle, which is three, the second pentagonal, twelve, is produced <math>\scriptstyle{\color{blue}{9+3=12}}</math>. | |
| − | + | |style="text-align:right;"|כמו שתמצא כי מקבוץ המרבע השני שהוא תשעה עם המשלש הראשון שהוא שלשה יולד שנים עשר המחמש השני | |
| − | |style="text-align:right;"| | + | |- |
| + | |Indeed, one finds such property in all the measured shapes: | ||
| + | |style="text-align:right;"|והנה לכל {{#annot:term|303,2471|1yEx}}התמונות השעוריות{{#annotend:1yEx}} תמצא סגלה כזאת | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|הנה | + | *The hexagonal is produced by summing the pentagonal that is in its rank with the triangle that is in the preceding rank: |
| + | |style="text-align:right;"|כי הנה ה{{#annot:term|1669,1777|2GIl}}משושה{{#annotend:2GIl}} יתחדש מקבוץ המחמש אשר במדרגתו עם המשלש אשר הוא למטה ממנו במדרגה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::*The second hexagonal number = <math>\scriptstyle{\color{blue}{15=12+3}}</math> = the second pentagonal number + the first triangular number. |
| + | |style="text-align:right;"|כמו ה{{#annot:term|1669,1777|7rJH}}מששה{{#annotend:7rJH}} השני שהוא חמשה עשר אשר נולד משנים עשר המחמש השני עם שלשה המשלש הראשון | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::*The second heptagonal number = <math>\scriptstyle{\color{blue}{18=15+3}}</math> = the second hexagonal number + the first triangular number. |
| + | |style="text-align:right;"|וכן ה{{#annot:term|1775,1778|cpzV}}משבע{{#annotend:cpzV}} השני שהוא שמנה עשר יתחדש מהמששה השני שהוא חמשה עשר ומשלשה המשלש הראשון | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::And so on. |
| + | |style="text-align:right;"|ועל הדרך הזה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *The pentagonal numbers are produced by taking the squares that are in their rank, and adding to the squares half their sides as many times as the number of the ranks: |
| + | |style="text-align:right;"|וכבר יולדו ה{{#annot:term|1672,87|FMzG}}מחמשים{{#annotend:FMzG}} בלקיחת המרבעים אשר במדרגתם וכפי מספר המדרגות כל כך פעמים נוסיף על המרבע ההוא מספר חצי צלעו | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::*The first square number + half its side = [<math>\scriptstyle{\color{blue}{4+1=5}}</math>] = the first pentagonal number. |
| + | |style="text-align:right;"|דמיון זה לקחנו ארבעה המרבע הראשון והוספנו עליו חצי צלעו ועלה חמשה המחמש הראשון | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::*The second square number + twice the half of its side = [<math>\scriptstyle{\color{blue}{9+3=12}}</math>] = the second pentagonal number. |
| + | |style="text-align:right;"|ולקחנו תשעה המרבע השני וקבצנו עמו שני פעמים חצי צלעו ועלה שנים עשר המחמש השני | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::*The third square number + three times the half of its side = [<math>\scriptstyle{\color{blue}{16+6=22}}</math>] = the third pentagonal number. |
| + | |style="text-align:right;"|ולקחנו ששה עשר המרבע השלישי והוספנו עליו שלשה פעמים חצי צלעו ועלה שנים ועשרים המחמש השלישי | ||
| + | |- | ||
| + | |This property is also related to all the measured shapes: | ||
| + | |style="text-align:right;"|וכן הסגלה הזאת דבקה לכל התמונות השעוריות | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *For the hexagonal one takes the side, instead of half the side. |
| + | |style="text-align:right;"|אמנם למשושה תמורת חצי צלע נקח צלע | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *For the heptagonal the side and its half instead of this. |
| + | |style="text-align:right;"|ולמשבע תמורת זה צלע וחצי | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | *For the octagonal - two sides |
| + | |style="text-align:right;"|ול{{#annot:term|1776,1779|uuX7}}משמן{{#annotend:uuX7}} שתי צלעות | ||
|- | |- | ||
| − | | | + | |So on, adding half the side by half the side, then multiplying the result by the number of the ranks. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכן תמיד בתוספת חצי צלע חצי צלע אח"כ בהכפלת המגיע במספר המדרגות |
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | ::For example: |
| + | ::[hexagonal number = <math>\scriptstyle{\color{red}{\left(n+1\right)^2+\left(n+1\right)\sdot n}}</math>] | ||
| + | ::*The first square number + its side = [<math>\scriptstyle{\color{blue}{4+2=6}}</math>] = the first hexagonal number. | ||
| + | |style="text-align:right;"|משל זה לקחנו ארבעה המרבע הראשון וקבצנו עמו צלעו פעם ועלה ששה המששה הראשון | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::*The second square number + twice its side = [<math>\scriptstyle{\color{blue}{9+6=15}}</math>] = the second hexagonal number. |
| + | |style="text-align:right;"|לקחנו תשעה המרבע השני וקבצנו עמו צלעו שתי פעמים ועלה חמשה עשר המששה השני | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::[heptagonal number = <math>\scriptstyle{\color{red}{\left(n+1\right)^2+\left[\left(n+1\right)\sdot n\left(1+\frac{1}{2}\right)\right]}}</math>] |
| + | ::*The first square number + one and a half times its side = [<math>\scriptstyle{\color{blue}{4+3=7}}</math>] = the first heptagonal number. | ||
| + | |style="text-align:right;"|עוד לקחנו ארבע המרבע הראשון וקבצנו עמו צלעו וחצי צלעו פעם ועלה שבעה המשבע הראשון | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::*The second square number + [three] times its side = [<math>\scriptstyle{\color{red}{9+9=18}}</math>] = the second heptagonal number. |
| + | |style="text-align:right;"|לקחנו תשעה המרבע השני ועמו קבצנו שני פעמים צלעו וחצי צלעו ועלה שנים עשר המשבע השני ועל הדרך הזה כלם | ||
| + | |- | ||
| + | |All these are composed from triangles. | ||
| + | |style="text-align:right;"|וכבר התחברו אלה כלם מהמשלשי‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*The square is composed from two triangles. |
| + | |style="text-align:right;"|וכמו שהמרבע יתרכב משני משלשים | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*The pentagon is composed from two triangles. |
| + | |style="text-align:right;"|כמו כן המחמש יתרכב משלשה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*The hexagon - from four. |
| + | |style="text-align:right;"|והמששה מארבעה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*The heptagon - from five. |
| + | |style="text-align:right;"|והמשבע מחמשה | ||
| + | |- | ||
| + | |In a way that is similar to the composition of the squares. | ||
| + | |style="text-align:right;"|על דרך דומה לדרך חבור המרבעים | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::For example: |
| + | ::*The second pentagonal, which is 12, consists of twice the first triangle and once the second triangle. | ||
| + | |style="text-align:right;"|ויהיה דרך משל המחמש השני שהוא י"ב מרכב מהמשלש הראשון שתי פעמים והמשלש השני פעם | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::*The third pentagonal [consists of] twice the second triangle and once the third triangle. |
| − | + | |style="text-align:right;"|והמחמש השלישי מהמשלש השני שתי פעמים והמשלש השלישי פעם | |
| − | |||
|- | |- | ||
| | | | ||
| − | + | ::*The second hexagonal, which is 15, consists of the second pentagonal that consists of three triangles, plus the first triangle that precedes it. | |
| − | = | + | |style="text-align:right;"|וכן המששה השני שהוא ט"ו מורכב מהמחמש השני המורכב משלשה משלשי' ומהמשלש הראשון אשר למטה ממנו |
| − | + | |- | |
| | | | ||
| + | ::Hence, it is possible to say that one is also in the rank of a triangle. | ||
| + | |style="text-align:right;"|ואפשר לומר לפי זה שיהיה האחד ג"כ כן במדרגת משלש | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | ::For the first pentagonal consists of four and one [<math>\scriptstyle{\color{blue}{4+1=5}}</math>] |
| + | |style="text-align:right;"|וזה כי המחמש הראשון הוא מורכב מארבעה ואחד | ||
|- | |- | ||
| | | | ||
| − | + | ::Also, every measured square is divided into two triangles, therefore, it is proper that one is instead of one triangle. | |
| − | + | |style="text-align:right;"|וכל מרבע שעוריי יתחלק לשני משלשים ולזה מן הנכון שיהיה האחד כמו כן במקום משלש אחד | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | *Every hexagon is a triangle, but not vice versa. |
| + | |style="text-align:right;"|וכל מששה משלש ולא יתהפך | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::*<math>\scriptstyle{\color{blue}{6}}</math> = the first hexagonal number = the second triangular number. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כי הנה הששה מששה ראשון הוא המשלש השני |
|- | |- | ||
| | | | ||
| − | ::* | + | ::*<math>\scriptstyle{\color{blue}{15}}</math> = the second hexagonal number = the fourth triangular number. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וט"ו המששה השני הוא המשלש הרביעי |
|- | |- | ||
| | | | ||
| − | ::* | + | ::*<math>\scriptstyle{\color{blue}{28}}</math> = the third hexagonal number = the sixth triangular number. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכ"ח המששה השלישי הוא המשלש הששי |
|- | |- | ||
| | | | ||
| − | : | + | :Not every triangle is a hexagonal number. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואין כל משלש מששה |
|- | |- | ||
| | | | ||
| − | :: | + | ::Such as <math>\scriptstyle{\color{blue}{3;\;10;\;21}}</math>, which are triangles, but not hexagonal numbers. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כמו ג' י' וכ"א שהם משלשים ואינם מששים |
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | *Every triangular number whose position is an even number is not a hexagonal number. |
| + | |style="text-align:right;"|וכל משלש מספרו זוג הנה אין שתוף בינו ובין המששה‫<ref>marg. א"ב ר"ל מספר מדרגתו זוג בהתחיל מן האחד כמו ג' וי' שאינם מששים</ref> | ||
|- | |- | ||
| | | | ||
| − | : | + | *Finding the position of the triangle according to the hexagon: subtracting one from double the position of the hexagon [starting from one] and vice versa, adding one to the position of the triangle, then taking its half. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכאשר תרצה שתמצא מדרגת המשלש מהמששה הנה תגרע האחד מכפל מספר מדרגת המששה‫<ref>marg. כמו שתמצא ט"ו שהוא המששה השלישי הנה הוא המשלש החמישי וזה בהתחיל מן האחד</ref> והפוכו שתוסיף אחד על מספר מדרגת המשלש ותקח חציו |
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | *Every pentagonal number is half the product of the sum of the product of its position minus one, multiplied by the difference between the numbers from which the [pentagonal numbers] are produced, which is three, plus two, multiplied by the position of the pentagonal number. |
| + | :[pentagonal number = <math>\scriptstyle{\color{red}{\frac{1}{2}\sdot\left[\left[\left[3\sdot\left(n-1\right)\right]+2\right]\sdot n\right]}}</math>] | ||
| + | |style="text-align:right;"|וכל {{#annot:term|1672,87|qbh8}}מספר מחמש{{#annotend:qbh8}} הנה הוא חצי מה שיתקבץ מהכאת מספר חסר ממדרגתו באחד בתוספת אשר בין המספרים אשר יתילדו מהם‫<ref>marg.א"ב הם מספרי א'ד'ז'י' הנזכרי' למעלה שהתוספת ג' ג' ומה שבין שני מספרי א' ד' או ד' ז' או ז' י' הוא שנים על משך המספרי' הטבעיי'</ref> והוא שלשה נוסף עליו מה שבין שני מספרים מזה והוא שנים מוכה במספר מדרגתו מ{{#annot:term|1672,87|pedg}}המחמשים המספריים{{#annotend:pedg}} | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::For example: |
| − | |style="text-align:right;"| | + | :*<math>\scriptstyle{\color{blue}{\frac{1}{2}\sdot\left[\left[\left(3\sdot3\right)+2\right]\sdot4\right]=\frac{1}{2}\sdot\left[\left(9+2\right)\sdot4\right]=\frac{1}{2}\sdot\left(11\sdot4\right)=\frac{1}{2}\sdot44=22}}</math> |
| + | ::which is the fourth pentagonal number. | ||
| + | |style="text-align:right;"|המשל בזה כאשר תרצה שתדע המחמש הרביעי תכה שלשה בשלשה ויהיו תשעה ותוסיף עליהם שנים ויהיו אחד עשר תכה אותם בארבעה ויהיו ארבעים וארבעה תקח חצים ויהיו שנים ועשרים והוא המחמש הרביעי | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *Every pentagonal number is the same as the product of its position, beginning from one, by itself, plus the [product of] half its side by its position among the pentagonal numbers [without one]. |
| + | :[pentagonal number = <math>\scriptstyle{\color{red}{n^2+\left[\left(\frac{1}{2}\sdot n\right)\sdot\left(n-1\right)\right]}}</math>] | ||
| + | |style="text-align:right;"|וגם כן כי כל מחמש הנה הוא כמו הכאת מספר מדרגתו נמנית מן האחד בעצמו נוסף עליו חצי צלעו בתוספת מדרגתו במחמשים המספריים‫<ref>marg.א"ב ר"ל נוסף עליו הכאת חצי צלעו במדרגתו מן המחמשים</ref> | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::The same example [= the fourth pentagonal number]: |
| + | :*<math>\scriptstyle{\color{blue}{\left(4\sdot4\right)+\left[\left(\frac{1}{2}\sdot4\right)\sdot3\right]=16+\left(2\sdot3\right)=22}}</math> | ||
| + | |style="text-align:right;"|המשל בזה בשאלה הנזכרת שנכה ארבעה בארבעה לפי שהוא במדרגה הרביעית מן האחד ויהיו ששה עשר ותוסיף עליו חצי צלעו והוא שנים ושלשה מדרגות ויהיו שנים ועשרים‫<ref>marg. א"ב נראה שהוא בשלשה מדרגות ור"ל שנכה חצי הצלע והוא שנים בשלשה ויהיה ששה והם עם ששה עשר שנים ועשרים</ref> | ||
|- | |- | ||
| − | | | + | |After the pentagons the hexagons |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואחר המחמשים ה{{#annot:term|1669,1777|EUUb}}מששים{{#annotend:EUUb}} |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :They are composed from the numbers that are exceeding four by four. |
| + | |style="text-align:right;"|ויתחברו מקבוץ המספרים העודפים בארבעה ארבעה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Such as: <math>\scriptstyle{\color{blue}{1;\;5;\;9;\;13;\;17;\;21}}</math> |
| + | |style="text-align:right;"|כמו א' ה' ט' י"ג י"ז כ"א | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :As said for the pentagonal numbers. |
| + | |style="text-align:right;"|על הקש מה שנאמר במחמשים | ||
|- | |- | ||
| − | | | + | |Then the heptagons |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אח"כ ה{{#annot:term|1775,1778|hSin}}משבעים{{#annotend:hSin}} |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :They are composed from the numbers that are exceeding five by five. |
| + | |style="text-align:right;"|ויתחברו מקבוץ המספרים העודפים בחמשה חמשה | ||
|- | |- | ||
| − | | | + | |The same is applied to all of them. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכן תקיש בכלם |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *For every surface that succeeds the triangle, when added to the triangle, the surface that succeeds this [surface] by the number of sides, is generated. |
| + | |style="text-align:right;"|ונאמר שכל שטח אחר המשלש כאשר יחובר עם המשלש יתחדש השטח אשר ימשך לזה השטח במספר הצלעות | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Such as: |
| + | ::*The first triangular number, which is three, when summed with the second square, will be a pentagonal number. | ||
| + | |style="text-align:right;"|כמו המשלש הראשון והוא שלשה כשיתחבר עם המרבע השני יהיה מחמש | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::*When it [= the first triangular number] is summed with the second pentagonal, which is 12, it will be a hexagonal number, which is 15. |
| + | |style="text-align:right;"|וכאשר יתחבר עם המחמש השני והוא שנים עשר יהיה מששה והוא החמשה עשר | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::So on in this way. |
| + | |style="text-align:right;"|ועל זה הדרך | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *The excess of every surface over the preceding [is a triangle ?] |
| + | |style="text-align:right;"|ומותר כל שטח על אשר לפניו | ||
|- | |- | ||
| − | | | + | |? |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכבר נזדמן זה ולא יתהפך |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *For every perfect number, there is a triangle or hexagon [after] it. |
| + | |style="text-align:right;"|וכל מספר שלם הנה הוא לפניו מששה או משלש | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :By this, there is also a way for reaching the extraction of the ranks of the perfect numbers. |
| + | |style="text-align:right;"|ועוד יהיה מזה דרך נגיע בו אל הוצאת מדרגות המספרים השלמים גם כן | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :When you are told: the first perfect number, which triangle or hexagon is it? |
| + | |style="text-align:right;"|כי כאשר יאמר לך המספר השלם הראשון מאיזה מהמשלשים או מהמששים הוא | ||
|- | |- | ||
| | | | ||
| − | + | :Examine the arrangement known in particular: | |
| − | + | |style="text-align:right;"|הנה תעיין אל הסדור אשר ידעת אותו בזה האחד בפרט | |
| − | |||
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*The first even-times-even number, for which the known arrangement is noticed, is four: |
| + | |style="text-align:right;"|ותמצא הראשון שב{{#annot:term|1334,69|PhIH}}זוגי הזוגות{{#annotend:PhIH}} יבחן בו הסדור הידוע ארבעה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :::<math>\scriptstyle{\color{blue}{4-1=3}}</math> a prime number, appropriate for the procedure. |
| + | |style="text-align:right;"|וכאשר תגרע ממנו אחד ישאר שלשה מספר ראשון ונכון הוא לפעלתך | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :::<math>\scriptstyle{\color{blue}{\frac{1}{2}\sdot4=2}}</math> |
| + | |style="text-align:right;"|ותקח חציו והוא שנים | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::*the second hexagonal number and the third triangular number. |
| + | |style="text-align:right;"|ותאמר שהוא המששה השני והמשלש השלישי | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*[The even-times-even number] that follows four is eight: |
| + | |style="text-align:right;"|וימשך אל הארבעה שמנה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :::<math>\scriptstyle{\color{red}{8-1=7}}</math> prime numbe, appropriate for the requested [procedure] |
| + | |style="text-align:right;"|ותמצא השבעה כמו זה מספר ראשון ויתכן למבוקשך | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :::<math>\scriptstyle{\color{blue}{\frac{1}{2}\sdot8=4}}</math> |
| + | |style="text-align:right;"|ותחלק לחציים השמנה ויהיה ארבעה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::*the fourth hexagonal number and the seventh triangular number. |
| + | |style="text-align:right;"|ותאמר שהוא המששה הרביעי והמשלש השביעי | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*[The even-times-even number] that follows eight is sixteen: |
| + | |style="text-align:right;"|וימשך לשמנה ששה עשר | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :::<math>\scriptstyle{\color{blue}{16-1}}</math> composite number, not appropriate for the procedure |
| + | |style="text-align:right;"|וכאשר תגרע ממנו אחד ישאר מורכב והנה איננו נכון לפעלתך | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*[The even-times-even number] that follows sixteen is thirty two: |
| + | |style="text-align:right;"|וימשך אל הששה עשר שנים ושלשים | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|וכאשר | + | :::<math>\scriptstyle{\color{blue}{32-1}}</math> prime number, appropriate for the procedure |
| + | |style="text-align:right;"|וכאשר תגרע ממנו אחד ישאר ראשון והוא נכון לפעלתך | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :::<math>\scriptstyle{\color{blue}{\frac{1}{2}\sdot32=16}}</math> |
| + | |style="text-align:right;"|ותקח חציו והוא ששה עשר | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::*the 16th hexagonal number and the 31th triangular number. |
| + | |style="text-align:right;"|והנה תאמר המששה הששה עשר והמשלש האחד ושלשים | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::So on according to this rule. |
| + | |style="text-align:right;"|ועל זה ההקש | ||
|- | |- | ||
| − | | | + | |See the wonderful diagram, from which it is clear that all the geometric shapes are produced from the triangle and dissolved to it: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ראה צורה נפלאה יתבאר ממנה שכל התמונות מתילדי' מהמשלש ונתכים אליו |
| + | |} | ||
| + | |||
| + | {|class="wikitable" style="margin-left: auto; margin-right: auto; text-align:center;" | ||
|- | |- | ||
| − | | | + | |{{#annot:term|2110,1781|QsIS}}מעשרים{{#annotend:QsIS}}||{{#annot:term|2107,1780|liM4}}מתשעים{{#annotend:liM4}}||{{#annot:term|1776,1779|TqtO}}משמנים{{#annotend:TqtO}}||משבעים||מששים||מחמשים||מרבעים||משלשים |
| − | | | ||
|- | |- | ||
| − | | | + | |י||ט||ח||ז||ו||ה||ד||ג |
| − | | | ||
|- | |- | ||
| − | | | + | |כז||כד||כא||יח||טו||יב||ט||ו |
| − | | | ||
|- | |- | ||
| − | | | + | |נב||מו||מ||לד||כח||כב||יו||י |
| − | | | ||
|- | |- | ||
| − | | | + | |פה||עה||סה||נה||מה||לה||כה||טו |
| − | | | ||
|- | |- | ||
| − | | | + | |קכו||קיא||צו||פא||סו||נא||לו||כא |
| − | | | ||
|- | |- | ||
| − | | | + | |קעה||קנד||קלג||קיב||צא||ע||מט||כח |
| − | | | ||
|- | |- | ||
| − | | | + | |רלב||רד||קעו||קמח||קכ||צב||סד||לו |
| − | | | ||
|- | |- | ||
| − | | | + | |רצז||רסא||רכה||קפט||קנג||קיז||פא||מה |
| − | | | ||
|- | |- | ||
| − | | | + | |שע||שכה||רפ||רלה||קצ||קמה||ק||נה |
| − | |style="text-align: | + | |} |
| + | {|class="wikitable" style="color: blue; margin-left: auto; margin-right: auto; text-align:center;" | ||
| + | |decagon||nonagon||octagon||heptagon ||hexagon||pentagon||square||triangle | ||
|- | |- | ||
| − | | | + | |10||9||8||7||6||5||4||3 |
| − | | | ||
|- | |- | ||
| − | | | + | |27||24||21||18||15||12||9||6 |
| − | | | ||
|- | |- | ||
| − | | | + | |52||46||40||34||28||22||16||10 |
| − | | | ||
|- | |- | ||
| − | | | + | |85||75||65||54||45||35||25||15 |
| − | |style="text-align:right;"| | + | |- |
| + | |126||111||96||81||66||51||36||21 | ||
| + | |- | ||
| + | |175||154||133||112||91||70||49||28 | ||
| + | |- | ||
| + | |232||204||176||148||120||92||64||36 | ||
| + | |- | ||
| + | |297||261||225||189||153||117||81||45 | ||
| + | |- | ||
| + | |370||325||280||235||190||145||100||55 | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | :*When starting from 1, which is the first potential triangle, and adding it to 3, which is the first actual triangle, 4 is produced, which is the first actual square <math>\scriptstyle{\color{blue}{1+3=4}}</math>. | ||
| + | |style="text-align:right;"|וזה שאתה כשהתחלת מן האחד ראשון המשלשים בכח וצרפת אותו אל השלשה ראשון המשלשים בפעל נתילד מהם ארבעה ראשון המרבעים בפעל | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*<math>\scriptstyle{\color{blue}{3+6=9}}</math> the second square. |
| + | |style="text-align:right;"|וכאשר צרפת ג' עם ו' הנמשך לו נתילד מהם ט' המרבע השני | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :And so on. |
| + | |style="text-align:right;"|וכן תמיד | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*When returning to 1, which is the first potential triangle, and adding it to 4, 5 is produced, which is the first actual pentagon <math>\scriptstyle{\color{blue}{1+4=5}}</math>. |
| + | |style="text-align:right;"|אח"כ כשתשב אל האחד ראשון המשלשי' בכח וצרפתהו עם ד' ישוב ה' מחמש ראשון בפעל | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*<math>\scriptstyle{\color{blue}{3+9=12}}</math> the second pentagon. |
| + | |style="text-align:right;"|ובצרוף ג' לט' יתילד י"ב מחמש שני | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*<math>\scriptstyle{\color{blue}{6+16}}</math> the third pentagon. |
| + | |style="text-align:right;"|ומו' וי"ו מחמש שלישי | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*When returning to 1 and adding it to 5, the first actual hexagon is produced <math>\scriptstyle{\color{blue}{1+5}}</math>. |
| + | |style="text-align:right;"|וכשתשוב לאחד ותצרפהו עם ה' יוליד מששה ראשון בפעל | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*<math>\scriptstyle{\color{blue}{3+12}}</math> the second hexagon. |
| + | |style="text-align:right;"|וג' עם י"ב יוליד מששה שני | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :And so on. |
| + | |style="text-align:right;"|וכן תמיד | ||
|} | |} | ||
{| | {| | ||
| Line 3,583: | Line 4,497: | ||
| | | | ||
| − | === | + | ==== Solid Numbers ==== |
| − | |||
| − | |||
| − | |||
| | | | ||
| − | |||
|- | |- | ||
| − | | | + | |Now the solid numbers will be discussed. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<big>ונדבר</big> עתה ב{{#annot:term|1851,89|e9bf}}מספרים המוגשמים{{#annotend:e9bf}} |
|- | |- | ||
| | | | ||
| − | + | ===== Pyramids with Sharp Apex ===== | |
| − | |||
| | | | ||
| − | |||
|- | |- | ||
| − | | | + | |The first of them are the pyramids. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והראשונים שבהם ה{{#annot:term|1530,1785|CdXq}}מחדדים{{#annotend:CdXq}} |
|- | |- | ||
| − | | | + | |The pyramids are the most famous of the sharp solids, beginning from a broad base, then they do not cease from growing, until reaching the end of the sharp limit, the one. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וה{{#annot:term|1530,1097|Lvtr}}מחדדים{{#annotend:Lvtr}} והם הנודעים ב{{#annot:term|2109,2132|Pmc5}}מתלהבים{{#annotend:Pmc5}} הם אשר יתחילו מתושבת מרוחת אח"כ לא יסורו מלצמוח עד שיגיעו אל קצה הגבול מן החדות אל האחד |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *The first of them is that whose base is triangular. |
| + | |style="text-align:right;"|והראשון שבהם אשר תושבתו {{#annot:term|1527,85|SDvA}}משלשת{{#annotend:SDvA}} | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*The first of them is four, which is the first number that is linear, plane, and solid. |
| + | |style="text-align:right;"|והראשון מאלו הם הארבעה והנה הם המספר הראשון אשר הוא {{#annot:term|2101,2462|iEhP}}קויי{{#annotend:iEhP}} ו{{#annot:term|1880,83|VOd2}}שטחיי{{#annotend:VOd2}} ו{{#annot:term|1851,89|0lbJ}}מגשם{{#annotend:0lbJ}} | ||
| + | |- | ||
| + | |Their production: | ||
| + | :*[Triangular pyramids]: from summing the triangular numbers successively. | ||
| + | |style="text-align:right;"|ויתילד מחבור המשלשים על המשכם | ||
| + | |- | ||
| + | |?? | ||
| + | |style="text-align:right;"|הדרכה לחסרים מהם על המוסיף עד שיכלו אל האחד | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*Those whose base is four [sided]: from summing the square numbers according to this description. |
| + | |style="text-align:right;"|אח"כ אשר תושבתם ארבעה ויתילדו ומחבור המרבעים על זה התאר | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :In this way for those whose base is pentagonal and those whose base is hexagonal. |
| + | |style="text-align:right;"|ועל הדרך הזה אשר תושבתם {{#annot:term|1672,87|M8lz}}מחמשת{{#annotend:M8lz}} ואשר תושבתם {{#annot:term|1669,1777|edA4}}משששת{{#annotend:edA4}} | ||
| + | |- | ||
| + | |?? | ||
| + | |style="text-align:right;"|וכל {{#annot:term|1569,83|3wnz}}מספר מושטח{{#annotend:3wnz}} יתרכבו ממנו יקרא ואשר יחסר מצדו יקרא | ||
| + | |- | ||
| + | |Their production: | ||
| + | |style="text-align:right;"|והתילדותם | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *Those whose base is a triangle |
| + | |style="text-align:right;"|אם אשר תושבתו משלש | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*When the first triangle is added to one, so that they are <math>\scriptstyle{\color{blue}{4}}</math> = The first pyramid. |
| + | |style="text-align:right;"|הנה כאשר יצורף אל האחד המשלש הראשון ויהיו ארבעה והנה הוא המחדד הראשון | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*When the second triangle is further added to these, so that they are <math>\scriptstyle{\color{blue}{10}}</math> = The second pyramid of this type. |
| + | |style="text-align:right;"|וכאשר יצורף עם אלו עוד המשלש השני ויהיו עשרה יעלה המחדד השני מזה המין | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *Those whose base is a square |
| + | |style="text-align:right;"|ואמנם אשר תושבתם מרבע | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*The first of them = 1 + the first square |
| + | |style="text-align:right;"|הנה הראשון שבהם מן האחד והמרבע הראשון | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*The second = 1 + the first square + the second square |
| + | |style="text-align:right;"|והשני אליו מן האחד והמרבע הראשון והמרבע השני | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :The same applies to those whose base is a pentagon, a hexagon, and the other. |
| + | |style="text-align:right;"|ואשר תושבתם מחמש ומששה וזולת זה הנה הם על זה ההקש | ||
|- | |- | ||
| + | |The property of the angles and the sides and their number is according to the rule of the geometric shapes that have magnitude. | ||
| + | |style="text-align:right;"|ואמנם ענין הזויות והצלעות ומספרם הנה הוא על הקש התמונות בעלות הגודל | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ===== Prisms ===== | ||
| + | |||
| | | | ||
| − | |style="text-align:right;"| | + | |- |
| + | |The prism is also among the numerical solid shapes. | ||
| + | |style="text-align:right;"|וה{{#annot:term|1100,2112|aqI8}}מתגורר{{#annotend:aqI8}} גם כן מהתמונות המספריות המוגשמות | ||
| + | |- | ||
| + | |It is [produced] from multiplying the triangles and their relation to one another. | ||
| + | |style="text-align:right;"|והוא מהכפלת המשלשים ודבקות קצתם בקצת | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*Six is the first prism, which is produced from the first triangle, it has three sides, each of which is four-sided, and two sides, each of which is a triangle. |
| + | |style="text-align:right;"|והששה ה{{#annot:term|1100,2111|9vMo}}מגורר{{#annotend:9vMo}} הראשון שיתילד מן המשלש הראשון לו שלשה צלעות כל צלע בעל ארבעה ושתי צלעות כל צלע משלש | ||
| + | |- | ||
| + | |? | ||
| + | |style="text-align:right;"|אמנם הצלעות במספרם | ||
|- | |- | ||
| − | | | + | |The solid shapes encompassed by six surfaces are necessarily: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואמנם התמונות המגשמות אשר יקיפו בהם ששה שטחים הנה לא ימלטו |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *either their length, breadth, and depth are equal, such as <math>\scriptstyle{\color{blue}{10\times10\times10}}</math> - called cube. |
| + | |style="text-align:right;"|אם שיהיו ארכם ורחבם ועמקם שוים ויהיו כמו עשר בעשר אח"כ בעשר ויקרא מעקב | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *Or two of their dimensions are equal and one dimension is different. |
| + | |style="text-align:right;"|ואם שיהיו שני קטרים מהם שוים וקטר מתחלף | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*When the different dimension is smaller - it is called brick. |
| + | |style="text-align:right;"|וכאשר יהיה הקטר המתחלף יותר קטן יקרא לבניי | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*When it is greater - it is called beam |
| + | |style="text-align:right;"|וכאשר יהיה יותר גדול יקרא עמודיי | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *If the smaller surface is a circle - it is called circular |
| + | |style="text-align:right;"|אם יהיה מושטחו הקטן עגלה יקרא מעגל | ||
|- | |- | ||
| − | | | + | |?? |
| − | |style="text-align:right;"|אח"כ | + | |style="text-align:right;"|כמו חמשה בחמשה אח"כ ביותר מחמשה |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|באשר היה שיהיו השלשה מתחלפים הנה יקרא כפי זאת הצורה |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*Example for a brick: <math>\scriptstyle{\color{blue}{4\times4\times3}}</math> |
| + | |style="text-align:right;"|ודמיון ה{{#annot:term|2114,94|Ium0}}לבניי{{#annotend:Ium0}} ארבעה בארבעה אח"כ בשלשה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*Example for a beam: <math>\scriptstyle{\color{blue}{4\times4\times5}}</math> |
| + | |style="text-align:right;"|ודמיון ה{{#annot:term|2102,2133|h7OA}}עמודיי{{#annotend:h7OA}} הארבעה בארבעה אח"כ בחמשה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ודמיון שלשה בארבעה בחמשה או בשמנה |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ומנהגם שיקראו המספר אשר ישוב כשהוכה בעצמו בעצמו אח"כ מה שנתקבץ כעצמו ועל הדרך הזה מספר עגול כמו בס' החמשה והששה |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*<math>\scriptstyle{\color{blue}{5\times5=25}}</math> |
| + | |style="text-align:right;"|כי החמשה בעצמו חמשה ועשרים | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{5\times5\times5=125}}</math> |
| + | |style="text-align:right;"|אח"כ בחמשה מאה ועשרים וחמשה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*<math>\scriptstyle{\color{blue}{6\times6=36}}</math> |
| + | |style="text-align:right;"|והששה בעצמו ששה ושלשים | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{6\times6\times6=216}}</math> |
| + | |style="text-align:right;"|אח"כ בששה מאתים וששה עשר | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|יש מן האנשים מי שיקראו משטחו עגלה ומעוגל ומעקבו כדור וכדוריי |
| + | |} | ||
| + | {| | ||
|- | |- | ||
| | | | ||
| − | + | ||
| + | ===== Cube Numbers ===== | ||
| + | |||
| + | | | ||
|- | |- | ||
| − | | | + | |The properties of the cube that should be investigated and what is are already known in the book of ''The Elements'': |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואשר ראוי שנחקור מעניני המעקב וכבר נודע ממנו כלל בספר היסודות |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *Among the properties of the cube: the root of every number, when multiplied by its consecutive, then by its preceding, then [the root] is added to the product, [the sum] is equal to [the cube]. |
| + | :<math>\scriptstyle\left[n\sdot\left(n+1\right)\sdot\left(n-1\right)\right]+n=n^3</math> | ||
| + | |style="text-align:right;"|ומסגלות המעקב שעקב כל מספר כאשר הוכה באשר ימשך אליו אח"כ באשר לפניו אח"כ יתוסף אשר לפניו על מה שיתקבץ יהיה שוה לו‫<ref>marg. א"ב המשל בזה שנרצה לדעת מעקב ג' הנה נכה ג' בד' ויהיה י"ב ונכה י"ב בשנים ויהיו כ"ד נוסיף עליו ג' ויהיה כ"ז יהיה שוה למעקבו וירצה באמרו אח"כ יתוסף אשר לפניו אשר לפני אשר ימשך אליו</ref> | ||
|- | |- | ||
| − | | | + | |Their production is when the successive odds are arranged, beginning from the one, then summed according to the rank, thus the cubes are produced successively. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אמנם התילדותם הנה כאשר יסודרו הנפרדים הנמשכים מתחילים מן האחד ואח"כ יקובצו כפי המדרגה הנה יתילדו המעקבים על המשכם |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::For example: 1; 3; 5; 7; 9; 11; 13 |
| + | |style="text-align:right;"|המשל בזה שיסודרו אחד שלשה חמשה שבעה תשעה אחד עשר שלשה עשר | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::*1 is a cube |
| + | |style="text-align:right;"|הנה האחד מעקב | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::*3 is on the second rank, hence it is necessary to sum two terms: <math>\scriptstyle{\color{blue}{3+5=8}}</math> which is a cube. |
| + | |style="text-align:right;"|ואחריו השלשה והוא במדרגה השנית הנה יחויב שתקבץ שתי מדרגות ותקבץ השלשה והחמשה והנה זה שמנה והוא מעקב | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::*7 is on the third rank, hence it is necessary to sum three terms: <math>\scriptstyle{\color{blue}{7+9+11=27}}</math> which is the third cube. |
| + | |style="text-align:right;"|ואחריהם השבעה והוא במדרגה השלישית והנה יחויב שתקבץ שלשה מדרגות והנה יהיו שבעה תשעה אחד עשר וכלל זה שבעה ועשרים והוא המעקב השלישי | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::And according to this rule. |
| + | |style="text-align:right;"|ועל זה המנהג | ||
|- | |- | ||
| − | | | + | |When wishing to know the first odd number, from which a known cube is composed, take the number of the rank of the cube from the first number of the cubes, and it is one less than the number of the terms, |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכאשר תרצה לדעת הנפרד הראשון שנתרכב ממנו המעקב הידוע הנה תקח מספר מדרגת המעקב מהמספר הראשון שבמעקבים והנה יהיה זה חסר מן הראשון באחד |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::For example: the third cube, if [one is] the first, there are three [cubes], and if [not] it is the second, so there are two. |
| + | |style="text-align:right;"|ויהיה דמיון שני אלו במעקב השלישי אם הראשון הנה הוא שלשה ואם השני הנה הוא שנים | ||
|- | |- | ||
| − | | | + | |Subtract the second from the square of the first, |
| − | + | |style="text-align:right;"|ותגרע השני ממרבע הראשון | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::As subtracting 2 from 9 and it is the first odd number from which the third cube is summed, which is 7. |
| − | |style="text-align:right;"|כמו | + | |style="text-align:right;"|כמו שתגרע הנה השנים מתשעה והנה הוא הנפרד הראשון אשר ממנו חבור המעקב השלישי וזה הוא שבעה |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Then, adding [2] to [9] and it is eleven, which is the last odd number from which the [third cube] is summed. |
| + | |style="text-align:right;"|אח"כ תוסיף אותו עליו והנה יהיה אחד עשר והוא הנפרד האחרון אשר ממנו הרכבתו | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Thus, it is composed from them and from what is between them. |
| + | |style="text-align:right;"|ונתרכב מהם וממה שביניהם‫<ref>marg. א"ב משל זה נרצה לדעת המספר הנפרד הראשון שנתרכב ממנו המעקב השלישי שהוא כ"ז הנה תקח מספר מדרגת המעקב הזה מהמספר הראשון שבמעקבים ויהיה ב' וזה חסר ממספר מהמדרגות הראשון שהיה ג' באחד כי כשנתחיל למנות מן האחד יהיו שלשה וכשנתחיל מראשון המספרים המעקבים יהיו שנים וכן תמיד ונגרע ג' שהוא מספר המדרגות השני ממרבע ג' שהוא מספר המדרגות השלישי והוא ט' וישארו ז' והוא הנפרד הראשון אשר ממנו חבור מעקב כ"ז אח"כ נוסיף ב' על המרבע הנזכר ויהיו י"א והוא המספר האחרון משני אלו ממה שביניהם נתרכב כ"ז</ref> | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *<math>\scriptstyle{\color{blue}{4;\;5;\;6;\;9}}</math> are always recurring in the units of their cubes, this is the instruction of the units of the root of the cube. |
| + | |style="text-align:right;"|והארבעה והחמשה והששה והתשעה חוזרים במעקביהם תמיד אחדים והנה יהיה זה הוראה על אחדי העקב | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Such as: |
| + | ::*<math>\scriptstyle{\color{blue}{4\times4\times4=64}}</math> | ||
| + | |style="text-align:right;"|כמו ארבעה בארבעה אח"כ בארבעה ויהיו ארבעה וששים | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::*<math>\scriptstyle{\color{blue}{9\times9\times9=729}}</math> |
| + | |style="text-align:right;"|והתשעה בתשעה אח"כ בתשעה והוא שבע מאות ותשעה ועשרים | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*The root of the cube of two is always with eight. |
| + | |style="text-align:right;"|אמנם עקב השנים הנה הוא בשמנה תמיד | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*The root of the cube of eight is always with two. |
| + | |style="text-align:right;"|ועקב השמנה הנה הוא בשנים תמיד | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*The root of the cube of seven is always with three. |
| + | |style="text-align:right;"|ועקב השבעה בשלשה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*The root of the cube of three is always with seven. |
| + | |style="text-align:right;"|ועקב השלשה בשבעה תמיד | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *cube × cube = cube |
| + | *cube ÷ cube = cube | ||
| + | |style="text-align:right;"|והכאת המעקב במעקב וחלוקתו עליו מעקב | ||
|- | |- | ||
| − | | | + | |?? |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והכאת מרבע שני מספרים במרבע מספר אחד יחסים יחס שני מעקבים |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *[The difference] between two successive cubes is [equal to] the product of the smaller of these two cubes by the number that follows it and exceeds over it by one, then by three, plus one. |
| + | :[<math>\scriptstyle{\color{red}{\left(n+1\right)^3-n^3=\left[n\sdot\left(n+1\right)\sdot3\right]+1}}</math>] | ||
| + | |style="text-align:right;"|החלוק בין שני מעקבים הנמשכים הנה הוא הכאת היותר קטן שבשני המעקבים במספר אשר ימשך אליו ויוסיף עליו באחד כן בשלשה אחר כן תוסיף עליו אחד‫<ref>marg.א"ב ר"ל המספר אשר ימשך אליו והוא הוא המוסיף עליו באחד</ref> | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *For every cube, when its root is subtracted from it, the remainder has a sixth [= <math>\scriptstyle{\color{red}{n^3-n}}</math> divisible by 6] |
| + | |style="text-align:right;"|וכל מעקב יוסר ממנו עקבו הנה יהיה לנשאר שתות שלם | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *Every cube minus one is counted by its root minus one [= <math>\scriptstyle{\color{red}{n^3-1}}</math> divisible by <math>\scriptstyle{\color{red}{n-1}}</math>] |
| + | |style="text-align:right;"|וכל מעקב זולת אחד הנה ימנה אותו עקבו זולת אחד | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *For every cube, its half and its double are not cubes [= <math>\scriptstyle{\color{red}{\frac{1}{2}\sdot\left(n^3\right);\;2\sdot\left(n^3\right)}}</math> are not cubes] |
| + | |style="text-align:right;"|וכל מעקב הנה חציו וכפלו בלתי מעקב | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *For every cube, when one is added to it and the product of the triangular number in its position by six, it is always the cube of its successive number. |
| + | :[<math>\scriptstyle{\color{red}{n^3+1+6\sdot\left[\frac{1}{2}\sdot\left[n\sdot\left(n+1\right)\right]\right]=\left(n+1\right)^3}}</math>] | ||
| + | |style="text-align:right;"|וכל מעקב יחובר אליו האחד והכאת המשלש אשר במדרגתו בששה תמיד הנה הוא המעקב אשר ימשך אליו | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :The cubes can be produced from these. |
| + | |style="text-align:right;"|והנה אפשר שיתילדו מאלו המעקבים | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *Among the properties of the cubes: their check [= modulo], according to the arithmetical procedure of the people of India, is either one, or eight, or nine. |
| + | |style="text-align:right;"|ומסגלות המעקבים שבחינתם אשר כפי מעשה המספר אשר לאנשי הודו יהיה אם אחד ואם שמנה ואם תשעה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*If it is one: the units of the sides are either one, or four, or seven. |
| + | |style="text-align:right;"|ואם היה אחד הנה אחדי הצלעות אחד או ארבעה או שבעה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*If it is eight: they are either eight, or two, or five. |
| + | |style="text-align:right;"|ואם היה שמנה הנה הוא שמנה או שנים או חמשה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*If it is nine: they are either three, or six, or nine. |
| + | |style="text-align:right;"|ואם היה תשעה הנה הוא שלשה או ששה או תשעה | ||
| + | |} | ||
| + | {| | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | |
| + | ===== Heteromecic and square numbers ===== | ||
| + | |||
| + | | | ||
| + | |- | ||
| + | |The numbers that have sides are divided: | ||
| + | |style="text-align:right;"|<big>וכבר</big> יתחלקו בעלי הצלעות מהמספר | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *Some of them are of the "same" length |
| + | |style="text-align:right;"|ויאמר שמהם מה שהוא הוא הוא הארך‫<ref>marg.ר"ל הוהיי הארך שמספר הצלעות שוה והם המרבעים</ref> | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *Some of the are of "other" length [= heteromecic number]. |
| + | |style="text-align:right;"|ומהם מה שהוא {{#annot:term|2115,1772|XsrB}}זולתיי הארך{{#annotend:XsrB}} | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *Some of them are of different length, which is when its length and its breadth differ by whatever is greater than one [= scalene number]. |
| + | |style="text-align:right;"|ומהם מה שהוא נבדל הארך והנה הוא אשר ההתחלפות בין ארכו ורחבו במה שהוא למעלה מאחד יהיה איך שיהיה | ||
| + | |- | ||
| + | |The custom of those who discuss arithmetic to bring in this place a discussion that is derived from the people of demonstration, which is very similar to a rhetorical and poetical discussion, but we reject this and we demonstrate it by calling the heteromecic a heteromecic number. | ||
| + | |style="text-align:right;"|ומנהג המדברים במלאכת המספר שיביאו בזה המקום ובמה שירוץ מרוצתו דבור יוצא ממנהג אנשי המופת ודומה הרבה למאמר ההלציים והשיריים ונברח מזה ונוכיח עליו בקריאתם הזולתיי הארך בזולתיי הארך | ||
| + | |- | ||
| + | |It seems that the first "otherness" that falls between the numbers is in one, and it is the basis of the heterogeneity and its beginning, as it is the basis of the number itself. | ||
| + | |style="text-align:right;"|וידמה שיהיה ה{{#annot:term|1772,2199|jTnG}}זולתיות{{#annotend:jTnG}} הראשון שיפול בין המספר והמספר הוא באחד ויהיה הוא שרש ההתחלפות והתחלתו כמו שהוא שרש המספר עצמו | ||
| + | |- | ||
| + | |The heteromecic numbers are those [whose length and breadth] differ by one. | ||
| + | |style="text-align:right;"|ויהיו המספרי' הזולתיים הארך הם המשתנים באחד | ||
| + | |- | ||
| + | |The heteromecic surfaces are those that are encompassed by two heteromecic sides. | ||
| + | |style="text-align:right;"|והשטחים הזולתיים הם אשר יקיפו בהם שתי צלעות זולתיות | ||
|- | |- | ||
| − | | | + | |Setting a table: |
| − | |style="text-align:right;"| | + | :*Arranging the odd numbers successively, starting from one, on one line. |
| + | |style="text-align:right;"|וכאשר נרשום לוח יסודרו בו הנפרדים על המשכם מתחילים מן האחד בשורה אחת | ||
|- | |- | ||
| | | | ||
| − | : | + | :*The even numbers successively, starting from two, on another line. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והזוגות על המשכם מתחילים מן השנים בשורה אחרת |
|- | |- | ||
| − | | | + | |As is known, by summing the odd numbers, the square numbers are produced. |
| − | + | |style="text-align:right;"|הנה יולדו מקבוץ הנפרדים כפי מה שידעתו אותו המספרים המרבעים | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| − | | | + | |By summing the even numbers, the heteromecic numbers are produced. |
| − | + | |style="text-align:right;"|ויולדו מקבוץ הזוגות {{#annot:term|2115,1772|HRNQ}}המספרים הזולתיים הארך{{#annotend:HRNQ}} | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| − | | | + | |The "same" squares are produced from the odd numbers and the "others" from the even numbers as a necessary result. |
| − | + | |style="text-align:right;"|ויתילדו מן הנפרדים המרבעים ההויים ומן הזוגות הזולתיים כפי החיוב | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| − | | | + | |The pythagoreans start from this place, in a way that has no perfection. |
| − | + | |style="text-align:right;"|ויתחילו הפיתגוריים מזה המקום בדרך אין שלמות לו | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| − | | | + | |When summing the squares once again in a line, attributes and properties are seen from the position of the two lines: |
| − | + | |style="text-align:right;"|וכאשר תקבץ המרבעים פעם שנית בשורה יראה משכונת שתי השורות ענינים וסגלות | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | :*The first heteromecic numbers to the first of the square numbers = double ratio | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ומהם שאתה תמצא ראשון {{#annot:term|2115,1772|DBjI}}הזולתיים{{#annotend:DBjI}} על יחס הכפל מראשון המרבעים והוא המוסיף דמיון |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*The second to the second = sesquialter ratio |
| + | |style="text-align:right;"|והשני אצל השני על יחס המוסיף חצי | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*The third to the third = sesquitertian ratio |
| + | |style="text-align:right;"|והשלישי אצל השלישי על יחס המוסיף שליש | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :So on for all according to the succession of the numbers and the positions. |
| + | |style="text-align:right;"|ועל הדרך הזה כלם על דרך המספרים והמדרגות | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*The fourth = [sesquiquartan ratio] |
| + | |style="text-align:right;"|המשל בזה שהוא לרביעי רובע | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*The fifth = [sesquiquintan ratio] |
| + | |style="text-align:right;"|ולחמישי חומש | ||
|- | |- | ||
| − | | | + | |One finds the exceedance according to the ratio of the natural numbers: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ותמצא ההעדף על יחס המספרים הטבעיים |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*The excess of the first position is one. |
| + | |style="text-align:right;"|כי הנה העדף המדרגה הראשונה אחד | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*The excess of the second position is two. |
| + | |style="text-align:right;"|והעדף המדרגה השנית שנים | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :And so on. |
| + | |style="text-align:right;"|ועל הדרך הזה | ||
|- | |- | ||
| − | | | + | |When one is omitted and the numbers are set vis-a-vis, the ratio is like this: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכאשר ישמט האחד ויהיה הנכחיות בין מה שהוא מספר יבא היחס כמו זה |
|- | |- | ||
| − | | | + | |The excess is [now] in what was [previously] deficient: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואמנם התוספת מצד מה שהיה ממנו החסרון |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*<math>\scriptstyle{\color{blue}{4:2}}</math> double ratio |
| + | |style="text-align:right;"|יהיה הארבעה לשנים על יחס הכפל | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*<math>\scriptstyle{\color{blue}{9:6}}</math> sesquialter ratio |
| + | |style="text-align:right;"|והתשעה לששה על יחס המוסיף חצי | ||
|- | |- | ||
| | | | ||
| − | :* | + | :*<math>\scriptstyle{\color{blue}{16:12}}</math> sesquitertian ratio |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והששה עשר לשנים עשר על יחס המוסיף שליש |
|- | |- | ||
| | | | ||
| − | : | + | :And so on. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ועל הדרך הזה |
|- | |- | ||
| − | | | + | |The difference is according to the ratio of the natural numbers, starting from two. |
| − | + | |style="text-align:right;"|ויהיה החלוף על יחס המספרים הטבעיים מתחילים מן השנים | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| − | | | + | |Arranging the first of the "others" after the first square, starting from one, then their second after the second square. |
| − | + | |style="text-align:right;"|אח"כ כאשר תסדר ראשון הזולתיים אחר המרבע הראשון מתחיל מן האחד והשני להם אחר המרבע השני | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| − | | | + | |The ratio itself is compound: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|הנה יבא זה היחס בעצמו מחבר |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*<math>\scriptstyle{\color{blue}{2:1=4:2}}</math> |
| + | |style="text-align:right;"|ויהיה יחס השנים אל האחד כיחס הארבעה אל השנים | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|כיחס | + | :*<math>\scriptstyle{\color{blue}{6:4=9:6}}</math> sesquialter ratio |
| + | |style="text-align:right;"|ויהיה יחס הששה אל הארבעה כיחס התשעה אל הששה והוא יחס המוסיף חצי | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכבר יסודרו תמיד ותהיינה השתי קצוות מבלי יחס מספרי כשיקובץ עם כפל האמצעי מרבע |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אח"כ כשתקבץ השתי שורות על סדורם |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ותתחיל הנפרדים מן האחד יתילדו מהם המספרים המשלשים על סדורם |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *For whichever has sides, when its side is subtracted from it, the "other", which is next to it by subtraction, is produced. |
| + | :<math>\scriptstyle{\color{red}{n^2-n=n\sdot\left(n-1\right)}}</math> | ||
| + | |style="text-align:right;"|ותמצא כל בעל צלע כאשר יחוסר ממנו צלעו יתילד הזולתי אשר הוא בשכונתו מצד החסרון | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *When its side is added to it, the "other", which is next to it by addition, is produced. |
| + | :<math>\scriptstyle{\color{red}{n^2+n=n\sdot\left(n+1\right)}}</math> | ||
| + | |style="text-align:right;"|וכאשר תוסיף עליו צלעו יתילד הזולתיי אשר הוא בשכונתו מצד התוספת | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכאשר תגיע‫<ref>marg. צ"ע כמו שורה אחת</ref> צלע המעקב ממנו ישאר צלעותיו ממנו |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכאשר תקח {{#annot:term|1569,83|OOPk}}מושטח{{#annotend:OOPk}} בין שני מרבעים תמצא המרבע הראשון יקח ממנו יחס והמרבע השני יחס אחר אמנם ישובו אל היחסים הנמשכים מתחילים מן הכפל אח"כ הדמיון והחצי |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ועל הדרך הזה אמרו הנה הנפרדים יתנו סבת ה{{#annot:term|86,2514|eAaB}}הוא הוא{{#annotend:eAaB}} ולקח ה[ההויים] |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ולכן יתילדו מהם המרבעים והמעקבים וימצאו במדרגות הנפרדי' מרבע ולא ימצאו במדרגות הזוגות כלל |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|נשלם המאמר השלישי מן אלארתמיטיקי שבח לאל |
| + | |} | ||
| + | {| | ||
|- | |- | ||
| | | | ||
| − | + | ||
| − | + | === Proportions === | |
| | | | ||
| − | |||
|- | |- | ||
| − | | | + | |It is a common practice to mention at this point the ratios, their types and their properties. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכבר פשט המנהג לזכור בזה המקום היחסים ומיניהם וסגלותיהם |
|- | |- | ||
| − | | | + | |Some people established many types of relations, reaching up to twenty types and some were satisfied with ten, which were translated ancients. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ומן האנשים מי שיחדש להתיחסויות פנים רבים ויגיעו בהם אל עשרים פנים ומהם שנסתפקו על עשרה והוא המועתק מהקדמון |
|- | |- | ||
| − | | | + | |The intention is to be satisfied with mentioning these ten and not tending to bring everything they brought and to mention everything they said, because this has no perfection. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ומכונתי שאסתפק בזכר אלו העשרה ועם ההסתפקות בהם לא תטה נפשי אל הבאת כל מה שהביאוהו וזכרון כל מה שאמרו אותו כי זה ממה שאין שלמות לו |
|- | |- | ||
| − | | | + | |It should be known that these are the examined relations, and most of their realization is in the diversity between them and the different properties whose diversity is in one order: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואתה ראוי שתדע שאלו ההתיחסויות הנבחני' ורוב הגעתם במה שיש ביניהם ההתחלפות והענינים המתחלפים אשר ירוץ התחלפותם על סדר אחד |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*Continuous, such as: <math>\scriptstyle{\color{blue}{A:B=B:C}}</math> |
| + | |style="text-align:right;"|אם מתדבק כמו יחס א' אל ב' כמו ב' אל ג' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*Discontinuous, such as: <math>\scriptstyle{\color{blue}{A:B=C:D}}</math> |
| + | |style="text-align:right;"|או מתחלק כמו יחס א' אל ב' כמו ג' אל ד' | ||
|- | |- | ||
| − | | | + | |Either their similarity and the uniqueness of their arrangement is in the quantity of themselves or in their quantity according to other. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אם שיהיה התדמותם והתיחדות סדורם בכמות נפשם או כמותם אצל זולתם |
|- | |- | ||
| − | | | + | |This is the root and the examined. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וזה הוא השרש והנבחן |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *The similarity of the diversity of the numbers by their quantity itself: as when the excess of this over that is equal to the excess of third over the fourth. |
| + | :<math>\scriptstyle a_2-a_1=a_4-a_3</math> | ||
| + | |style="text-align:right;"|והתדמות חלוף המספרים בכמות נפשם הוא כמו שיהיה תוספת זה על זה שוה לתוספת השלישי על הרביעי | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Such as: the excess of six over four, and ten over eight, or four over two.<math>\scriptstyle{\color{blue}{6-4=10-8=4-2}}</math> |
| + | |style="text-align:right;"|כמו תוספת הששה על הארבעה והעשרה על השמנה או הארבעה על השנים | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :These are the arithmetic relations |
| + | |style="text-align:right;"|ואלו הם {{#annot:term|2098,1769|v8ed}}ההתיחסויות המספריים{{#annotend:v8ed}} | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *The similarity of the diversity of the numbers by their quantity in relation to the others: as when the added quantity of this over that is equal to the quantity of third over the fourth, or when the quantity of the different by the different from it is one. |
| + | :<math>\scriptstyle a_1:a_2=a_3:a_4</math> | ||
| + | |style="text-align:right;"|והדמות חלופי המספרים בכמותם אצל זולתם הרי הוא כמו שיהיה כמות תוספת זה על זה כמו כמות זה השלישי על הרביעי או יהיה כמות זה המתחלף אצל המתחלף אליו אחד | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Such as the property of <math>\scriptstyle{\color{blue}{4:2}}</math> is as the property of <math>\scriptstyle{\color{blue}{10:{\color{red}{5}}}}</math> |
| + | |style="text-align:right;"|כמו ענין הארבעה אצל השנים בהתחלפות הוא כמו ענין העשרה אצל הששה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :This is the geometric relation |
| + | |style="text-align:right;"|וזה הוא {{#annot:term|2097,1770|6SJH}}ההתיחסות התשברתיי{{#annotend:6SJH}} | ||
|- | |- | ||
| − | | | + | |These two are in essence two roots |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ושני אלו באמתות שני שרשים |
|- | |- | ||
| − | | | + | |When someone examines the property of difference of the relative quantity and the difference of the numerical quantity in the arithmetic proportion [and the geometric proportion], they are found different and no harmony is found at all: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אמנם כאשר יבחן אי ענין חלוף הכמות הצרופיי בחלוף הכמות המספריי ב{{#annot:term|2098,1769|SoRu}}התיחסות המספריי{{#annotend:SoRu}} וענין חלוף הכמות הצרופיי נמצאו מתחלפות והנה לא ימצא הנה הסכמה כלל |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::For example: |
| + | ::*Setting a geometric ratio, such as <math>\scriptstyle{\color{blue}{4;\;6;\;9}}</math> | ||
| + | |style="text-align:right;"|המשל בזה שנניח {{#annot:term|2097,1770|CuIW}}יחס תשברתיי{{#annotend:CuIW}} כמו ארבעה ששה תשעה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :::The relative quantities are similar, but the quantities of the numbers themselves are not similar, for the difference in one [pair] is two, and in the other [pair] is three. |
| + | |style="text-align:right;"|והנה הכמות המצטרף בהם מתדמה והכמות אשר למספר בעצמו בלתי מתדמה כי החלוף באחד מהם שנים ובאחר שלשה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::*Setting an arithmetic ratio, such as <math>\scriptstyle{\color{blue}{4;\;6;\;8}}</math> |
| + | |style="text-align:right;"|והנה נניח {{#annot:term|2098,1769|4kcu}}יחס מספריי{{#annotend:4kcu}} כמו ארבעה וששה ושמנה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :::The differences of the quantities themselves are equal, but the difference of the quantities in relation are not similar: |
| + | |style="text-align:right;"|ותמצא חלוף הכמו' בעצמו שוה וחלוף הכמות בהקש בלתי מתדמה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :::*<math>\scriptstyle{\color{blue}{6:4}}</math> sesquialter ratio |
| + | |style="text-align:right;"|אבל יהיה ששה לארבעה מוסיף חצי | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :::*<math>\scriptstyle{\color{blue}{8:6}}</math> sesquitertian ratio |
| + | |style="text-align:right;"|ושמנה לששה אינו מוסיף כי אם בשליש | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::The two ratios are always continuous, but the greater ratio among them is between the two smaller numbers and the smaller [ratio] of them is between the two greater numbers. |
| + | |style="text-align:right;"|וימצאו שני היחסים תמיד נמשכים אבל היחס הגדול מהם בין שני המספרים היותר קטנים והקטן מהם בין שני המספרים היותר גדולים | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Their ratio is one in this sense that one seeks for numbers whose mutual relation is a relation that sets the two ratios between them continuous, and sets the greater between the greater and the smaller between the smaller. |
| + | |style="text-align:right;"|ויחסם מזה הענין אחד והוא שתבקש מספרים חבורם חבור ישים השני יחסים אשר ביניהם נמשכים וישים הגדול בין הגדולים והקטן בין הקטנים | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *One ratio is found according to this description, such as the ratio between <math>\scriptstyle{\color{blue}{6;\;4;\;3}}</math>, and it is called harmonic, since the benefit from the observation of this proportion is fulfilled in the art of composition, which is music, as will be known in its place. |
| + | |style="text-align:right;"|והנה ימצא יחס אחד על זה התאר כמו יחס מה שבין הששה והארבעה והשלשה ויקרא חבוריי לפי שהתועלת בהשגחת אמצעי זה ההתיחסות אמנם יפול במלאכת החבור והוא המוסיקי כפי מה שתדעהו במקומו | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :It is possible that it is called harmonic [lit. compositional], since the ratio of the extremes is composed from the ratio of the excesses, as is known. |
| + | |style="text-align:right;"|וכבר יעבר שיהיה נקרא חבוריי לפי שיחס הקצוות מחובר מיחס ה{{#annot:term|1508,877|TRPO}}מותרות{{#annotend:TRPO}} כפי מה שתדע | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :It has necessarily the property that the ratio of the excess of the greater over the mean to the excess of the mean over the smaller is the ratio of the greater extreme to the smaller [extreme]. |
| + | :<math>\scriptstyle\left(a_3-a_2\right):\left(a_2-a_1\right)=a_3:a_1</math> | ||
| + | |style="text-align:right;"|ויתחיב לו סגלה שיחס {{#annot:term|1508,877|s6XK}}מותר{{#annotend:s6XK}} הגדול על האמצעי אל מותר האמצעי על הקטן הוא יחס ה{{#annot:term|1902,2120|K7OM}}קצה{{#annotend:K7OM}} הגדול אל הקטן | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Such as: <math>\scriptstyle{\color{blue}{\left(a_3-a_2\right):\left(a_2-a_1\right)=\left(6-4\right):\left({\color{red}{4-3}}\right)=2:1={\color{red}{3:6}}=a_3:a_1}}</math> |
| + | |style="text-align:right;"|כמו יחס השנים והוא מותר הששה על הארבעה אל אחד אשר הוא מותר השלשה על השנים‫<ref>marg. א"ב צ"ע ונראה סיומו כיחס ששה אל שלשה ואפשר שהוא כך אשר הוא מותר הארבעה על השלשה כיחס ששה אל שלשה</ref> | ||
|- | |- | ||
| − | | | + | |From this property that is necessary for this ratio, they observed the examination of the excess of the related terms, and gradually moved from them to other relations and proportions that are useful from the aspect of perfection of the division or multiplication of the ratio. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אח"כ הם התבוננו מזאת הסגולה אשר נתחיבה לזה היחס לבחינת התיחסויות מותר הגבולים המתיחסים והלכו בהדרגה מהם אל התיחסויות ואמצעים אחרים אמנם יועילו מצד השלמות חלוקת היחס או רבויו |
|- | |- | ||
| − | | | + | |We will begin with each relation and proportion and say a brief statement about them figuratively: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ונתחיל ב{{#annot:term|1278,564|DoDM}}התיחסות{{#annotend:DoDM}} התיחסות ואמצעי אמצעי ונאמר בהם דבור קצר על דרך העברה |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *The geometric mean is the root of the product of the extremes - it is the root of the product of the two extremes one by the other. |
| + | :<math>\scriptstyle a_2=\sqrt{a_1\sdot a_3}</math> | ||
| + | |style="text-align:right;"|אם האמצעי התשברתיי הנה אמנם יהיה גדר הכאת הקצוות והנה יהיה גדר מה שיתקבץ משתי הקצוות אחד מהם באחר | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :This is already known from another place. |
| + | |style="text-align:right;"|וכבר ידעת זה ממקום אחר | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *It is known that when there are two means instead of the [one] mean, the product of one of them by the other is as the product of one of the two extremes by the other. |
| + | :<math>\scriptstyle a_2\sdot a_3=a_1\sdot a_4</math> | ||
| + | |style="text-align:right;"|וידעת כי כשהיה תמורת האמצעי שני אמצעיים הנה הכאת אחד מהם באחר כהכאת אחת משתי הקצוות באחרת | ||
| + | |- | ||
| + | |This leads you to the investigation of the mean: | ||
| + | |style="text-align:right;"|וזה יורה אליך אל דרישת האמצעי | ||
| + | |- | ||
| + | |It is known in this investigation that the geometric relations are added three by three in the gradual progress of the successive "other" with the successive squares. | ||
| + | |style="text-align:right;"|וידעת כי בזאת החקירה שאלו {{#annot:term|2097,1770|SC4F}}ההתיחסויות התשברתיות{{#annotend:SC4F}} יתחברו שלשה שלשה בהדרגת הזולתיים הנמשכים עם המרבעים הנמשכי' | ||
| + | |- | ||
| + | |You know from other places that every two squares may apply these properties. | ||
| + | |style="text-align:right;"|וכבר ידעת גם כן ממקומות אחרים שכל שני מרבעים אפשר שיפלו הודעת אלו הענינים | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *The arithmetic relation and proportion: whether in one or in ten [terms], you will find them continuous in this proportion as mentioned above. |
| + | |style="text-align:right;"|אמנם ההתיחסות והאמצעי המספרי באחד או בעשרה והנה שם תמצא אותם מתדבקים באמצעי זה ממה שכבר קדם לך | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :You know the property of the succession of the ratio and the multiplication of the ratio, which is that when half the sum of the two extremes is subtracted, as known, from the square of the mean, it is as the square of the difference. <math>\scriptstyle a_2^2-\frac{1}{2}\sdot\left(a_3+a_1\right)=\left(a_3-a_1\right)^2</math> |
| + | |style="text-align:right;"|וידעת ענין המשך היחס וכפילת היחס שלהם והוא שילקח חצי מקבץ השתי קצוות כפי מה שידעת ממרבע האמצעי בכמו מרבע המותר | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כמו שהכאת השנים בששה הוא השנים בעצמו |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *The harmonic relation and proportion: you already know its opposition to the arithmetic [proportion] in what is found opposed to it. |
| + | |style="text-align:right;"|אמנם ההתיחסות והאמצעי החבוריי כבר ידעת התנגדותם למספרי במה שימצא ההתנגדות בהם | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*The extraction of the mean is by multiplying the difference between the greater and the smaller by the smaller, dividing [the product] by their sum, then adding [the quotient] to the smaller and the result is the mean <math>\scriptstyle a_2=\frac{a_1\sdot\left(a_3-a_1\right)}{a_1+a_3}+a_1</math> |
| + | |style="text-align:right;"|והוצאת האמצעי בשנכה החלוף אשר בין הגדול והקטן בקטן ונחלק על מקובצם ונוסיף אותו על הקטן ויצא האמצעי | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Such as: <math>\scriptstyle{\color{blue}{a_2=\frac{3\sdot\left(6-3\right)}{3+6}+3=\frac{9}{3+6}+3=1+3=4}}</math> |
| + | |style="text-align:right;"|כמו שיהיה תשעה ותחלק על מקובץ השלשה והששה והנה יצא אחד ותוסיף אותו על השלשה ויהיו ארבעה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכאשר יהיה אצלך האמצעי והגדול ותרצה שתמצא הקטן תעיין אל מותר מה שביניהם כמה הוא מן האמצעי כשנחלק עליו האמצעי פעם אחרת והנה מה שיצא נגרע אותו מן האמצעי |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכאשר יהיו הקטן והאמצעי ידועים אצלך ותרצה הגדול תחלק האמצעי על המותר ותגרע ממנו אח"כ תחלק עליו ומה שיצא תוסיף אותו על האמצעי |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :*Among the properties of this proportion is that the product of the sum of the two extremes by the mean is as double the product of one of the extremes by the other <math>\scriptstyle\left(a_1+a_3\right)\sdot a_2=2\sdot\left(a_1\sdot a_3\right)</math> |
| + | |style="text-align:right;"|ומסגלות זה ההתיחסות שהכאת מקובץ השני קצוות באמצעי כמו כפל אחת הפאות באחרת | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וג"כ הנה הכאת האמצעי בגדול כמו כפל האמצעי בקטן בשעור הכפלת אחת משתי הקצוות אל האחרת |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכבר חשבו אנשים שזה היחס אמנם נקרא חבוריי לפי שמותרותיו שחלוקותיו אינם בגבולים לבדם ולא במותרות לבדם אבל קצת בזה וקצת בזה וכאלו נפל בזה חבור וזה טורח |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכבר אמרו מה שהוא יותר חזק הטרח מזה |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אמנם ההתיחסויות אשר אחר אלו הנה מהם שלשה נודעו ראשונה ומהם ארבעה נודעו שנית ומהם התיחסויות אין מכונתנו שנשגיח אליהם |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואלו הארבעה יודעו בשלישי והרביעי והחמישי והששי |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ויקרא הרביעי המתנגד לפי שהוא מנגד לחבוריי באשר הוא הושם בצד |
| + | |- | ||
| + | |The ratio of the excess of the mean over the smaller to the excess of the greater over the mean is as the ratio of the greater to the smaller | ||
| + | :<math>\scriptstyle\left(a_2-a_1\right):\left(a_3-a_2\right)=a_3:a_1</math> | ||
| + | |style="text-align:right;"|והנה יחס מותר האמצעי על הקטן ומותר הגדול על האמצעי כיחס הגדול אל הקטן | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::Such as: 15; 8; 6? |
| + | |style="text-align:right;"|כמו חמשה עשר ושמנה וששה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|הנה | + | |style="text-align:right;"|והוצאתם בהכאת המותר שבין שתי הקצוות הקטנו' בקטן והחלוקה על מקובצם והכפלת מה שיצא מן הגדול הנה הוא האמצעי סגלותיו שהכאת הגדול באמצעי כפל הכאת באמצעי התיחסות |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *The fifth proportion: the mean to the smaller is as the difference between the two smallers to the difference between the two greaters. |
| + | :<math>\scriptstyle a_2:a_1=\left(a_2-a_1\right):\left(a_3-a_2\right)</math> | ||
| + | |style="text-align:right;"|והאמצעי החמישי שיהיה האמצעי אצל הקטן כמו מותר השני קטנים אצל מותר השני גדולים | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ומספרם כאלו נגזר בזה התשברתיי |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ודרישת זה האמצעי שתוסיף הקטן על הגדול ותחלק מה שיתקבץ חלוקה תהיה הכאת אחד מהם באחר כהכאת הנשאר מהגדול אחר השלכת הקטן ממנו בקטן וזה נקל למי שידע היחס |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואם אפשר זה זה ואם לא הנה השאלה בטלה |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ומה שיצא יחוסר הקטן מהגדול ממנו ומה שנשאר הנה הוא האמצעי |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ומסגלותיו שהכאת הגדול באמצעי כפל הכאת הגדול בקטן |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|ואולם המין העשירי הנה הוא אשר יהיה בו יחס האמצעי אל הקטן כיחס מותר מה שבין שני הקצוות אל מותר מה שבין האמצעי והגדול כמו השלשה והחמשה והשמנה הנה אלו הם המינים העשרה ממיני המתיחסים שיספיק לנו זכרונם כי אין לאחד מהם מבא בחכמה זולתי מה שזכרנום לפנים ואולם הביאונום על צד שלמות החלוקה והמשכתה וספירת מה שיגיעו אליו צדי ההתיחסות נשלם זה והתהלה לאל ית' שמו | + | |style="text-align:right;"|ומזה שהאמצעי מהם בהתיחסות הכפליי נגדר לעולם וגדרו הקטן |
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|והקצה הגדול קטן ממקבץ הנשארים באחד | ||
| + | |- | ||
| + | | | ||
| + | *The sixth: the greater to the mean is as the difference between the two smallers [to the difference between the two greaters]. | ||
| + | :<math>\scriptstyle a_3:a_2=\left(a_2-a_1\right):\left(a_3-a_2\right)</math> | ||
| + | |style="text-align:right;"|הששי שיהיה הגדול אצל האמצעי כמו מותר השני קטנים | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ודמיון זה | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|והוצאת האמצעי כשנחסר הקטן מן הגדול כמה שיצטרך שיתוסף על הגדול והתוספת עד שיהיה הכאת זה בכל המקובץ מן השרש והשני תוספת כמו המושטח אשר שמר והנה מקובץ השני תוספות הוא האמצעי | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ואם נשבר הנה השאלה בטלה | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|וג"כ הנה אתה כאשר תגרע ותכפול גדרו ותגרע ממנו המוכה ראשונה בעצמו ומה שנשאר תוסיף אותו על הקטן | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|וכבר יחויב לו מן הסגלות שההתיחסות כשהיה על יחס הדמיון והחלק יהיה האמצעי נגדר | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|וכאשר יצטרף אליו גדרו יהיה מקבצו הקצה הגדול והקצה הקטן קטן ממנו | ||
| + | |- | ||
| + | | | ||
| + | *The seventh: the ratio of the difference between the two extremes to the difference between the two smallers is as the ratio of the greater to the smaller. | ||
| + | :<math>\scriptstyle\left(a_3-a_1\right):\left(a_2-a_1\right)=a_3:a_1</math> | ||
| + | |style="text-align:right;"|אמנם הארבעה אשר ידעת באחרונה הנה הראשון שבהם הוא השביעית שיהיה יחס המותר בין השתי קצוות אל המותר בין שני הקטנים כיחס הגדול אצל הקטן | ||
| + | |- | ||
| + | | | ||
| + | ::The example for this: 6; 8; 9. | ||
| + | |style="text-align:right;"|המשל בזה הששה והשמנה והתשעה | ||
| + | |- | ||
| + | | | ||
| + | :<math>\scriptstyle a_2=a_1+\frac{a_1\sdot\left(a_3-a_1\right)}{a_3}</math> | ||
| + | |style="text-align:right;"|והוצאת האמצעי שלו בהכאת הקטן במותר אשר בינו ובין הגדול וחלוקת המתקבץ על הגדול ותוספת היוצא על הקטן ומה שהגיע הנה הוא האמצעי | ||
| + | |- | ||
| + | | | ||
| + | *The eighth: the ratio of the greater to the smaller is as the ratio of the difference between the two extremes to the difference between the two greaters. | ||
| + | :<math>\scriptstyle a_3:a_1=\left(a_3-a_1\right):\left(a_3-a_2\right)</math> | ||
| + | |style="text-align:right;"|והשמיני שיהיה יחס הגדול אל הקטן כיחס מותר שתי הקצוות אל מותר השני גדולים | ||
| + | |- | ||
| + | | | ||
| + | ::The example for this: 6; 7; 9. | ||
| + | |style="text-align:right;"|המשל בזה ששה שבעה תשעה | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|והוא הפך השביעית | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|והוצאת האמצעי שלו הפך הוצאת זה האמצעי | ||
| + | |- | ||
| + | | | ||
| + | :<math>\scriptstyle a_2=a_3-\frac{a_1\sdot\left(a_3-a_1\right)}{a_3}</math> | ||
| + | |style="text-align:right;"|וזה בהכאת הקטן במותר אשר בין שתי הקצוות וחלוקת היוצא על הגדול ומה שיצא נגרע אותו מהגדול ומה שישאר הנה הוא האמצעי | ||
| + | |- | ||
| + | | | ||
| + | *The ninth: the ratio of the difference between the extremes to the difference between the two smallers is the ratio of the mean to the smaller. | ||
| + | :<math>\scriptstyle\left(a_3-a_1\right):\left(a_2-a_1\right)=a_2:a_1</math> | ||
| + | |style="text-align:right;"|התשיעי שיהיה יחס מותר הקצוות אל מותר השני קטנים יחס האמצעי אל הקטן | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|כמו הוצאת האמצעי שלו כשנגרע הקטן מהגדול ונחלק השני חלוקה תהיה אחד החלקים אל האחר כיחס האחר אל הקטן אם אפשר ונפיל החלק הראשון מהגדול ומה שישאר הנה הוא האמצעי | ||
| + | |- | ||
| + | | | ||
| + | :*<math>\scriptstyle\left(\frac{1}{2}\sdot a_1\right)+\sqrt{\left(\frac{1}{2}\sdot a_1\right)^2+\left[\left(a_3-a_1\right)\sdot a_1\right]}</math> | ||
| + | |style="text-align:right;"|ובידך שתקבץ הכאת המותר בקטן אל מרבע חצי הקטן ותקח גדרו ותוסיפהו על חצי הקטן | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|וזה ההתיחסות כאשר יהיה על יחס הדמיון והחלק יהיה הקצה הקטן מרבע תמיד | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|והתיחסות והאמצעי העשירי שיהיה יחס מותר השתי קצוות אל מותר השני גדולים | ||
| + | |- | ||
| + | | | ||
| + | :*<math>\scriptstyle\left(\frac{1}{2}\sdot a_3\right)+\sqrt{\left(\frac{1}{2}\sdot a_3\right)^2-\left[\left(a_3-a_1\right)\sdot a_1\right]}</math> | ||
| + | |style="text-align:right;"|כמו יחס האמצעי שלו שתקח מותר מה שבין הקצוות מוכה בקטן מחוסר ממרבע חצי הגדול ותקח גדר זה תוסיפהו על חצי הגדול | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|אלו צריכים בשתי קצוות עם התשברתיי תמיד ולא עם השביעי והשמיני ולא עם החבוריית אל שיהיה הגדול הפך הקטן כמו הרביעי אלא שיהיה הגדול גם כן הפך הקטן והתשבריית לא השמיני ימצאו עם החבוריים ולא עם הרביעי ולא עם השביעי ולא עם השמיני ולא עם התשיעי | ||
| + | |- | ||
| + | | | ||
| + | ::When 80 and 20 are given as two extremes: | ||
| + | |style="text-align:right;"|וכאשר הונחו לנו השמנים והעשרים שני {{#annot:term|1580,2120|tvnY}}גבולים{{#annotend:tvnY}} | ||
| + | |- | ||
| + | | | ||
| + | ::*50 is mean between them in the arithmetic proportion | ||
| + | |style="text-align:right;"|יהיו החמשים ביניהם {{#annot:term|2098,2123|KYdI}}אמצעי מספריי{{#annotend:KYdI}} | ||
| + | |- | ||
| + | | | ||
| + | ::*40 in the geometric proportion | ||
| + | |style="text-align:right;"|וארבעים {{#annot:term|2097,2122|a53U}}אמצעי תשברתיי{{#annotend:a53U}} | ||
| + | |- | ||
| + | | | ||
| + | ::*32 in the harmonic proportion | ||
| + | |style="text-align:right;"|ושנים ושלשים {{#annot:term|2095,2124|2PVB}}אמצעי חבוריי{{#annotend:2PVB}} | ||
| + | |- | ||
| + | | | ||
| + | ::*68 in the fourth proportion | ||
| + | |style="text-align:right;"|ושמנה וששים אמצעי רביעי | ||
| + | |- | ||
| + | | | ||
| + | ::*35 in the seventh proportion | ||
| + | |style="text-align:right;"|וחמשה ושלשים אמצעי שביעי | ||
| + | |- | ||
| + | | | ||
| + | ::*65 in the eighth proportion | ||
| + | |style="text-align:right;"|וחמשה וששים אמצעי שמיני | ||
| + | |- | ||
| + | |The fifth, the sixth, the ninth, and the tenth are extracted: | ||
| + | |style="text-align:right;"|וכבר הוצאו החמישי והששי והתשיעי והעשירי | ||
| + | |- | ||
| + | | | ||
| + | ::*The first terms of the fifth proportion are ? [<math>\scriptstyle a_1;\;a_2;\;a_3</math>] | ||
| + | |style="text-align:right;"|והנה נניח ראשון גבולי ההתיחסות החמישי והם | ||
| + | |- | ||
| + | | | ||
| + | ::*When one is subtracted from the smaller and added to the greater, they are ? [<math>\scriptstyle a_1-1;\;a_2;\;a_3+1</math>] and it is the sixth proportion. | ||
| + | |style="text-align:right;"|וכאשר חוסר מהקטן אחד ונוסף על הגדול היו והוא ההתיחסות הששי | ||
| + | |- | ||
| + | | | ||
| + | ::*When two is added to each of the terms, they are ? [<math>\scriptstyle a_1+2;\;a_2+2;\;a_3+2</math>] the ninth proportion is resulted. | ||
| + | |style="text-align:right;"|וכאשר נוסף על כל גבול שנים עד שיהיו יצא ההתיחסות התשיעי | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|וכאשר מן האמצעי אחר זה מה שאמרנוהו בחכמת הארתמיטיקי | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|וכבר הנחנו ענינים היה המנהג לחברם בזה המקום יוצאים מסדר המלאכה | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|וכבר אצל המעשה כמו החבור וההקבלה והקבוץ והחלוק ההוריי ומה שירוץ מרוצתם | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|והראוי בכמו אלו שיזכרו בענפים והנה נסתפק הנה על ההגעה הנזכרת ונקח בחכמת המוסיקי בע"ה | ||
| + | |- | ||
| + | |What is done with the number in relation and comparison: | ||
| + | |style="text-align:right;"|הנעשה במספר על זה ההצטרפות וההקש | ||
| + | |- | ||
| + | | | ||
| + | :When one of two numbers is related to the other, they are necessarily either equal or different. | ||
| + | |style="text-align:right;"|כשהוקש אחד משני מספרים אל האחר הנה לא ימלט מאשר יהיו שוים או מתחלפים | ||
| + | |- | ||
| + | | | ||
| + | :*If they are equal, the ratio between them is the ratio of equality and evenness. | ||
| + | |style="text-align:right;"|ואם היו שוים היה היחס ביניהם יחס השווי והיושר | ||
| + | |- | ||
| + | | | ||
| + | ::This ratio is conceived as the natural beginning of the other ratios, and to it every ratio is dissolved. | ||
| + | |style="text-align:right;"|וידמה שזה היחס הוא התחלה טבעית לשאר היחסים ושהוא אשר אליו יותך כל יחס | ||
| + | |- | ||
| + | | | ||
| + | ::The arrangement of this will be mentioned afterwards. | ||
| + | |style="text-align:right;"|ואיך יהיה זה בסדור הניחו בכלם נזכרהו עוד אחר זה | ||
| + | |- | ||
| + | | | ||
| + | *If they are different the ratio of the greater among them to the less is necessarily | ||
| + | :*The multiple ratio: as <math>\scriptstyle{\color{blue}{20:10;\;30:10}}</math> | ||
| + | |style="text-align:right;"|ואם היו מתחלפים לא ימלט אם שיהיה יחס היותר רב שבהם אל היותר מעט יחס הדמיונים כעשרים והשלשים אצל העשרה | ||
| + | |- | ||
| + | | | ||
| + | :*The superparticular ratio: as <math>\scriptstyle{\color{blue}{10:8}}</math> | ||
| + | |style="text-align:right;"|או {{#annot:term|1659,125|ssLz}}יחס הדמיון המוסיף חלק{{#annotend:ssLz}} כעשרה אצל השמנה | ||
| + | |- | ||
| + | | | ||
| + | :*The superpartient ratio: as <math>\scriptstyle{\color{blue}{10:6}}</math> | ||
| + | |style="text-align:right;"|או {{#annot:term|1660,128|VYdM}}יחס הדמיון המוסיף חלקים{{#annotend:VYdM}} כעשרה אצל הששה | ||
| + | |- | ||
| + | | | ||
| + | :*The multiple superparticular ratio: as <math>\scriptstyle{\color{blue}{10:3}}</math> | ||
| + | |style="text-align:right;"|או יחס הדמיונים המוסיף חלק כעשרה אצל השלשה | ||
| + | |- | ||
| + | | | ||
| + | :*The multiple superpartient ratio: as <math>\scriptstyle{\color{blue}{15:4}}</math> | ||
| + | |style="text-align:right;"|או יחס הדמיוני המוסיף חלקים כחמשה עשר אצל הארבעה | ||
| + | |- | ||
| + | |These are the categories of the numerical ratios when the greater is related to the smaller. | ||
| + | |style="text-align:right;"|ואלו הם חלוקות היחסים המספרים כאשר הוקש בהם היותר רב אל היותר מעט | ||
| + | |- | ||
| + | |When you know this, you know the property of the smaller to the greater. | ||
| + | |style="text-align:right;"|וכאשר ידעת זה ידעת ענין המעט אצל הרב | ||
| + | |- | ||
| + | |The less is called the opposite. | ||
| + | |style="text-align:right;"|ויקרא היותר מעט המקביל | ||
| + | |- | ||
| + | |The less in the sesquialter ratio, for example, is called the opposite to the sesquialter ratio. | ||
| + | |style="text-align:right;"|ויקרא היותר מעט ב{{#annot:sesquialter|125|f61F}}יחס הדמיון המוסיף חצי{{#annotend:f61F}} דרך משל המקביל לדמיון המוסיף חצי | ||
| + | |- | ||
| + | |In the sesquitertian ratio - the opposite to the sesquitertian ratio. | ||
| + | |style="text-align:right;"|וב{{#annot:sesquitertian|125|qENZ}}יחס הדמיון המוסיף שליש {{#annotend:qENZ}}המקביל לדמיון המוסיף שליש | ||
| + | |- | ||
| + | |And so on for the others. | ||
| + | |style="text-align:right;"|וכן בשאר | ||
| + | |- | ||
| + | |It is also [said?] about the less, as beneath so and so, it is said sub-super so and so sub-multiple so and so. | ||
| + | |style="text-align:right;"|וכבר יולץ ג"כ מן היותר מעט כאשר תחת פלוני ויאמר לו אשר תחת הדמיון המוסיף ככה או הדמיונים המוסיפים ככה | ||
| + | |- | ||
| + | |The arrangement of the production of all of these ratios is as mentioned. | ||
| + | |style="text-align:right;"|והסדור בהמצאת אלו היחסים כלם כפי מה שנזכרהו | ||
| + | |- | ||
| + | | | ||
| + | *The multiples: their property is clear. | ||
| + | |style="text-align:right;"|אם הדמיונים הנה הענין בהם מבואר | ||
| + | |- | ||
| + | | | ||
| + | :The first of them is the double, then follows the triple, after it the quadruple, after it the quintuple, and so on. | ||
| + | |style="text-align:right;"|והראשון שבהם השניי אחר ימשך אליו השלישיי אחריו הרביעיי אחריו החמישיי וכן תמיד | ||
| + | |- | ||
| + | | | ||
| + | :This ratio begins with two and follows the succession of the numbers endlessly. | ||
| + | |style="text-align:right;"|וזה היחס מתחיל מהשנים והולך על המשך המספר עד אין תכלית | ||
| + | |- | ||
| + | |The other ratios are found by taking the number by which the additional part or parts are denominated. | ||
| + | |style="text-align:right;"|אולם היחס אשר זולתו זה הנה אתה תמצאהו בשתקח המספר אשר בשמו יקרא החלק המוסיף או החלקים | ||
| + | |- | ||
| + | | | ||
| + | :*If wishing the superparticular ratio: adding this part to the number by which it is denominated, and the sum is the sought-after. | ||
| + | ::<math>\scriptstyle\left(1+\frac{1}{n}\right):1=\left[n+\left(\frac{1}{n}\sdot n\right)\right]:n</math> | ||
| + | |style="text-align:right;"|ואם תרצה {{#annot:term|1659,125|7uLZ}}הדמיון המוסיף חלק{{#annotend:7uLZ}} תוסיף זה החלק על זה המספר אשר יקרא בשמו ויתקבץ לך מה שתרצה | ||
| + | |- | ||
| + | | | ||
| + | ::For example: when wishing the sesquioctavian ratio: | ||
| + | |style="text-align:right;"|דמיון זה כשתרצה הדמיון ושמינית | ||
| + | |- | ||
| + | | | ||
| + | :::taking the number by which it is denominated, which is eight, adding to it its eighth, which is one, and their sum is nine. | ||
| + | :::<math>\scriptstyle{\color{blue}{8+\left(\frac{1}{8}\sdot8\right)=8+1=9}}</math> | ||
| + | |style="text-align:right;"|הנה תקח המספר אשר בשמו יקרא זה החלק והוא שמנה ותוסיף עליו שמיניתו וזה אחד ויתקבץ מהם תשעה | ||
| + | |- | ||
| + | | | ||
| + | ::Hence, <math>\scriptstyle{\color{blue}{9:8}}</math> is the sesquioctavian ratio. | ||
| + | |style="text-align:right;"|ויהיה יחס תשעה אל שמנה יחס הדמיון המוסיף שמינית | ||
| + | |- | ||
| + | | | ||
| + | :*Also if wishing the superbipartient ratio: | ||
| + | |style="text-align:right;"|וכן ג"כ אם תרצה יחס הדמיון המוסיף חלקים | ||
| + | |- | ||
| + | | | ||
| + | ::Such as: the superbiquintan ratio | ||
| + | |style="text-align:right;"|כמו המוסיף שתי חמישיות | ||
| + | |- | ||
| + | | | ||
| + | :::taking five, for [the ratio] is denominated by it, adding to it its two fifths, which is two, and their sum is seven. | ||
| + | :::<math>\scriptstyle{\color{blue}{5+\left(\frac{2}{5}\sdot5\right)=5+2=7}}</math> | ||
| + | |style="text-align:right;"|הנה תקח חמשה כי בשמו נקראים ותוסיף שני חמישיותיו והוא שנים ויתקבץ שבעה | ||
| + | |- | ||
| + | | | ||
| + | ::Hence, <math>\scriptstyle{\color{blue}{7:5}}</math> is the superbiquintan ratio. | ||
| + | |style="text-align:right;"|ויחס שבעה לחמשה יחס הדמיון המוסיף שתי חמישיות | ||
| + | |- | ||
| + | | | ||
| + | :*For the multiple superparticular or the multiple superpartient ratio: it is found by multiplying the number, by which the part or the parts are denominated, by the number of the given multiples, then adding the required part or parts of that number to the product, and the total is the sought-after. | ||
| + | ::<math>\scriptstyle\left(m+\frac{a}{n}\right):1=\left[\left(m\sdot n\right)+\left(\frac{a}{n}\sdot n\right)\right]:n</math> | ||
| + | |style="text-align:right;"|ואולם הדמיונים המוסיפים חלק או חלקים הנה תמצאהו כשתכפול המספר אשר בשמו יקראו החלק או החלקים כמספר הדמיונים המונחים ותוסיף על מה שיתקבץ החלק המבוקש מזה המספר או החלקים ויהיה הכלל כמבוקשך | ||
| + | |- | ||
| + | | | ||
| + | ::For example: if wishing the triple sesquiquintan ratio | ||
| + | |style="text-align:right;"|דמיון זה אם תרצה השלשה דמיונים המוסיף חומש | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{\left(5\sdot3\right)+\left(\frac{1}{5}\sdot5\right)=15+1=16}}</math> which is the greater. | ||
| + | |style="text-align:right;"|הנה תקח חמשה ותכפלהו במספר הדמיונים שהם שלשה ויעלה חמשה עשר ותוסיף על זה חומש החמשה שהוא אחד ויעלה ששה עשר והוא היותר רב | ||
| + | |- | ||
| + | | | ||
| + | :::Its ratio to five, which is the less <math>\scriptstyle{\color{blue}{16:5}}</math> is the triple sesquiquintan ratio. | ||
| + | |style="text-align:right;"|ויחסו אל החמשה שהוא היותר מעט יחס השלשה דמיונים המוסיף חומש | ||
| + | |- | ||
| + | | | ||
| + | :*For the multiple superpartient: | ||
| + | |style="text-align:right;"|וכן תעשה ביחס הדמיונים המוסיף חלקים | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{15+2=17}}</math> | ||
| + | |style="text-align:right;"|שתוסיף כאן שנים על החמשה עשר ויהיו שבעה עשר | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{17:5}}</math> is the triple superbiquintan ratio. | ||
| + | |style="text-align:right;"|ויחסם אל חמשה השלשה דמיונים המוסיף שתי חמישיות | ||
| + | |- | ||
| + | |When the parts are reduced to one part | ||
| + | |style="text-align:right;"|וכאשר ישובו החלקים אל חלק אחד | ||
| + | |- | ||
| + | | | ||
| + | ::Such as | ||
| + | ::*<math>\scriptstyle{\color{blue}{\frac{2}{6}=\frac{1}{3}}}</math> | ||
| + | |style="text-align:right;"|כמו שישובו השתי ששיות אל השליש | ||
| + | |- | ||
| + | | | ||
| + | ::*<math>\scriptstyle{\color{blue}{\frac{4}{8}=\frac{1}{2}}}</math> | ||
| + | |style="text-align:right;"|והארבעה שמיניות אל החצי | ||
| + | |- | ||
| + | | | ||
| + | ::And what is similar. | ||
| + | |style="text-align:right;"|ומה שדומה לזה | ||
| + | |- | ||
| + | |Their rule is according to the rule of what they are reduced to. | ||
| + | |style="text-align:right;"|הנה משפטם בסדור משפט מה שישובו אליו | ||
| + | |- | ||
| + | |Also, if the superparticular or the superpartient involves fraction of fraction: | ||
| + | |style="text-align:right;"|וג"כ אם יהיו המוסיף על הדמיון או הדמיונים חלק חלק | ||
| + | |- | ||
| + | | | ||
| + | ::Such as | ||
| + | ::*<math>\scriptstyle{\color{blue}{\frac{1}{6}\sdot\frac{1}{6}}}</math> | ||
| + | |style="text-align:right;"|כמו שתות השתות | ||
| + | |- | ||
| + | | | ||
| + | ::*<math>\scriptstyle{\color{blue}{\frac{1}{5}\sdot\frac{1}{5}}}</math> | ||
| + | |style="text-align:right;"|וחומש החומש | ||
| + | |- | ||
| + | | | ||
| + | ::*<math>\scriptstyle{\color{blue}{\frac{1}{3}\sdot\frac{1}{10}}}</math> | ||
| + | |style="text-align:right;"|או כשליש העשור | ||
| + | |- | ||
| + | | | ||
| + | ::*<math>\scriptstyle{\color{blue}{\frac{1}{5}\sdot\frac{1}{7}}}</math> | ||
| + | |style="text-align:right;"|וחומש השביע | ||
| + | |- | ||
| + | | | ||
| + | ::And what is more subtle than this. | ||
| + | |style="text-align:right;"|ומה שהוא יותר דק מזה | ||
| + | |- | ||
| + | | | ||
| + | ::*<math>\scriptstyle{\color{blue}{\frac{1}{5}+\frac{1}{6}}}</math> | ||
| + | |style="text-align:right;"|או אם היה חומש ושתות | ||
| + | |- | ||
| + | | | ||
| + | ::*<math>\scriptstyle{\color{blue}{\frac{1}{3}+\frac{1}{4}}}</math> | ||
| + | |style="text-align:right;"|או שליש ורביע | ||
| + | |- | ||
| + | | | ||
| + | ::And what is similar. | ||
| + | |style="text-align:right;"|ומה שדומה לזה | ||
| + | |- | ||
| + | |All of them are reduced to one part or parts. | ||
| + | |style="text-align:right;"|הנה אלה כלם ישובו אל החלק האחד או החלקים | ||
| + | |- | ||
| + | |The fraction of fraction is also a fraction: | ||
| + | |style="text-align:right;"|וזה כי חלק החלק הוא ג"כ חלק | ||
| + | |- | ||
| + | | | ||
| + | :*<math>\scriptstyle{\color{blue}{\frac{1}{6}\sdot\frac{1}{6}=\frac{1}{36}}}</math> | ||
| + | |style="text-align:right;"|כמו שתות השתות שהוא חלק מששה ושלשים | ||
| + | |- | ||
| + | | | ||
| + | :*<math>\scriptstyle{\color{blue}{\frac{1}{5}\sdot\frac{1}{5}=\frac{1}{25}}}</math> | ||
| + | |style="text-align:right;"|וחומש החומש שהוא חלק מחמשה ועשרים | ||
| + | |- | ||
| + | | | ||
| + | :*<math>\scriptstyle{\color{blue}{\frac{1}{3}\sdot\frac{1}{10}=\frac{1}{30}}}</math> | ||
| + | |style="text-align:right;"|וכן שליש העשור שהוא חלק משלשים | ||
| + | |- | ||
| + | | | ||
| + | :*<math>\scriptstyle{\color{blue}{\frac{1}{5}\sdot\frac{1}{7}=\frac{1}{35}}}</math> | ||
| + | |style="text-align:right;"|וחומש השביע שהוא חלק מחמשה ושלשים | ||
| + | |- | ||
| + | | | ||
| + | :*<math>\scriptstyle{\color{blue}{\frac{1}{3}+\frac{1}{4}=\frac{7}{12}}}</math> | ||
| + | |style="text-align:right;"|וכן השליש והרביע שבעה חלקים משנים עשר | ||
| + | |- | ||
| + | | | ||
| + | :*<math>\scriptstyle{\color{blue}{\frac{1}{5}+\frac{1}{6}=\frac{11}{30}}}</math> | ||
| + | |style="text-align:right;"|והחומש והשתות אחד עשר חלקים משלשים | ||
| + | |- | ||
| + | |The arrangement discussed concerning the reduction of these ratios to equality: | ||
| + | |style="text-align:right;"|ואולם הסדור אשר דברנו<ref>זכרנו</ref> בהשבת אלו היחסים אל יחס השווי | ||
| + | |- | ||
| + | | | ||
| + | :*Setting the ratio in three terms, so that the ratio of the greater to the mean is the ratio of the mean to the smaller <math>\scriptstyle a_3:a_2=a_2:a_1</math>. | ||
| + | |style="text-align:right;"|הוא שנניח זה היחס בשלשה {{#annot:term|1580,1578|rQ2L}}גבולים{{#annotend:rQ2L}} יהיה יחס הגדול מהם אל האמצעי הוא בעינו יחס האמצעי אל הקטן | ||
| + | |- | ||
| + | | | ||
| + | :*Subtracting always double the mean minus the smaller from the greater <math>\scriptstyle b_3=a_3-\left(2a_2-a_1\right)</math>. | ||
| + | |style="text-align:right;"|ונחסר לעולם מן הגדול כפל האמצעי מחוסר ממנו הקטן | ||
| + | |- | ||
| + | | | ||
| + | :*Subtracting the smaller from the mean <math>\scriptstyle b_2=a_2-a_1</math>. | ||
| + | |style="text-align:right;"|ונחסר מהאמצעי כמו הקטן | ||
| + | |- | ||
| + | | | ||
| + | :*Leaving the smaller as it is <math>\scriptstyle{\color{blue}{b_1=a_1}}</math>. | ||
| + | |style="text-align:right;"|ונניח הקטן כמו שהוא | ||
| + | |- | ||
| + | | | ||
| + | :If the three terms are equal - it is the required; if not doing again as was done at first, until they are equal. | ||
| + | |style="text-align:right;"|ואם יהיו שוים הגבולים השלשה הנה המבוקש ואם לא תשוב ותעשה כמו מה שעשינו ראשונה עד שיהיו שוים | ||
| + | |- | ||
| + | | | ||
| + | ::For instance: the quadruple ratio <math>\scriptstyle{\color{blue}{a_3=64;\;a_2=16;\;a_1=4}}</math> | ||
| + | |style="text-align:right;"|ודמיון זה ביחס הארבעה דמיוני שיהיה הגדול ס"ד והאמצעי י"ו והקטן ד' | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{b_3=a_3-\left(2a_2-a_1\right)=64-\left[\left(2\sdot16\right)-4\right]=64-28=36}}</math> | ||
| + | |style="text-align:right;"|הנה כשכפלנו האמצעי ונחסר ממנו הקטן נשארו כ"ח וכאשר נחסר זה מן הגדול נשארו ל"ו | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{b_2=a_2-a_1=16-4=12}}</math> | ||
| + | |style="text-align:right;"|וכשנחסר הקטן מן האמצעי נשארו י"ב | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{b_1=a_1}}</math> | ||
| + | |style="text-align:right;"|ונשאיר הקטן בענינו | ||
| + | |- | ||
| + | | | ||
| + | ::These three numbers <math>\scriptstyle{\color{blue}{b_3=36;\;b_2=12;\;b_1=4}}</math> are in the triple ratio. | ||
| + | |style="text-align:right;"|ויהיו אלו המספרים השלשה אשר הם ל"ו י"ב ד' על יחס השלשה דמיונים | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{c_3=b_3-\left[2b_2-b_1\right]=36-\left[\left(2\sdot12\right)-4\right]=36-20=16}}</math> | ||
| + | |style="text-align:right;"|וכשנשוב ונחסר ג"כ מן הל"ו כפל הי"ב מחוסר ממנו הד' וזה עשרים נשארו י"ו | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{c_2=b_2-b_1=12-4=8}}</math> | ||
| + | |style="text-align:right;"|אחר כן נחסר מן הי"ב הד' נשארו ח' | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{c_1=b_1=4}}</math> | ||
| + | |style="text-align:right;"|ונשאיר הד' כמו שהם | ||
| + | |- | ||
| + | | | ||
| + | ::The three numbers <math>\scriptstyle{\color{blue}{c_3=16;\;c_2=8;\;c_1=4}}</math> are in the double ratio. | ||
| + | |style="text-align:right;"|ויהיו המספרים השלשה אחר זה י"ו ח' ד' על יחס הכפל | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{d_3=c_3-\left[2c_2-c_1\right]=16-\left[\left(2\sdot8\right)-4\right]=16-12}}</math> | ||
| + | |style="text-align:right;"|וכשנשוב שלישית ונחסר כפל הח' מחוסר ממנו הד' וזה י"ב מן הי"ו | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{d_2=c_2-c_1=8-4=4}}</math> | ||
| + | |style="text-align:right;"|אח"כ נחסר הד' מן הח' | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{d_1=d_2=d_3=4}}</math> | ||
| + | |style="text-align:right;"|יהיו שוים הגבולים הנשארים ויהיה כל אחד מהם ארבעה | ||
| + | |- | ||
| + | | | ||
| + | :One sees how the quadruple is reduced to the triple that is simpler than it. | ||
| + | |style="text-align:right;"|ואתה רואה איך שב המרבע אל המשלש אשר הוא יותר פשוט ממנו | ||
| + | |- | ||
| + | | | ||
| + | :Then the triple [is reduced] to the double that is simpler than it. | ||
| + | |style="text-align:right;"|אח"כ המשלש אל השניי אשר הוא יותר פשוט ממנו | ||
| + | |- | ||
| + | | | ||
| + | :Then it is dissolved to equality. | ||
| + | |style="text-align:right;"|ואחר הותך אל יחס השווי | ||
| + | |- | ||
| + | | | ||
| + | ::Giving an example for superparticular ratio - the sesquialter ratio | ||
| + | |style="text-align:right;"|עוד נמשיל ביחס הדמיון וחלק ויהיה החצי | ||
| + | |- | ||
| + | | | ||
| + | ::Setting the three numbers in this ratio <math>\scriptstyle{\color{blue}{18;\;12;\;8}}</math> | ||
| + | |style="text-align:right;"|ונשים השלשה מספרים אשר הם על זה היחס י"ח י"ב ח' | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{b_1=a_3-\left[2a_2-a_1\right]=18-\left[\left(2\sdot12\right)-8\right]=18-16=2}}</math> | ||
| + | |style="text-align:right;"|כפלנו הי"ב וחסרנו ממנו ח' נשארו י"ו וחסרנו זה מי"ח ונשארו ב' | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{b_2=a_2-a_1=12-8=4}}</math> | ||
| + | |style="text-align:right;"|וכשנחסר ח' מן י"ב נשארו ד' | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{b_3=a_1=8}}</math> | ||
| + | |style="text-align:right;"|ונניח ח' כמו שהוא | ||
| + | |- | ||
| + | | | ||
| + | ::The three terms <math>\scriptstyle{\color{blue}{2;\;4;\;8}}</math> are in the double ratio. | ||
| + | |style="text-align:right;"|ויהיה השלשה גבולים ב'ד'ח' על יחס הכפל | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{c_3=b_3-\left[2b_2-b_1\right]=8-\left[\left(2\sdot4\right)-2\right]=8-6=2}}</math> | ||
| + | |style="text-align:right;"|ונשוב ונחסר מהח' שהוא הגדול כפל האמצעי שהוא ד' מחוסר ממנו הקטן שהוא ב' וזה ו' נשארו מהגדול ב' | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{c_2=b_2-b_1=4-2=2}}</math> | ||
| + | |style="text-align:right;"|וכשנחסר מהאמצעי ב' נשאר גם כן ב' | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{c_1=b_1=2}}</math> | ||
| + | |style="text-align:right;"|ונשאיר הקטן ב' כמו שהוא וזה יחס השווי | ||
| + | |- | ||
| + | | | ||
| + | :One sees also how the superparticular ratio is reduced to the equality. | ||
| + | |style="text-align:right;"|ואתה רואה ג"כ איך שב יחס הדמיון והחלק אל יחס השווי | ||
| + | |- | ||
| + | | | ||
| + | :How it is reduced first to the double ratio, then this ratio is dissolved to the equality. | ||
| + | |style="text-align:right;"|ואיך יותך ראשונה אל יחס הכפל השניי ואחר יותך זה יחס אל יחס השווי | ||
| + | |- | ||
| + | |The types of proportions: | ||
| + | |style="text-align:right;"|ומיני המתיחסים | ||
| + | |- | ||
| + | | | ||
| + | *Either from the aspect of continuity, in which the mean is shared between the two extremes, taken in relation, one time succeeding and one time preceding. | ||
| + | |style="text-align:right;"|אם שיהיו על צד הדבקות והם אשר יהיה האמצעי משותף בין שתי הקצוות ילקח בהתיחסות פעם נמשך ופעם קודם | ||
| + | |- | ||
| + | | | ||
| + | :Its terms are always three, no more and no less | ||
| + | |style="text-align:right;"|וגבוליו תמיד שלשה לא פחות ולא יתר | ||
| + | |- | ||
| + | | | ||
| + | ::As saying the ratio of A to B as the ratio of B to D <math>\scriptstyle{\color{blue}{A:B=B:D}}</math> | ||
| + | |style="text-align:right;"|כאמרנו יחס א' אל ב' כיחס ב' אל ד' | ||
| + | |- | ||
| + | | | ||
| + | *Or from the aspect of the difference, in which there is no one shared mean, but two means. | ||
| + | |style="text-align:right;"|ואם שיהיה על צד ההבדל ולא יהיה בזה אמצעי אחד משותף אבל אמצעיים | ||
| + | |- | ||
| + | | | ||
| + | ::As saying the ratio of A to B as the ratio of D to C <math>\scriptstyle{\color{blue}{A:B=D:C}}</math> | ||
| + | |style="text-align:right;"|כאמרנו יחס א' אל ב' כיחס ד' אל ח' | ||
| + | |- | ||
| + | | | ||
| + | :The means in this ratio are always more than three. | ||
| + | |style="text-align:right;"|והגבולים בזה היחס תמיד יותר משלשה | ||
| + | |- | ||
| + | |The types of proportions are numerous, of them Nicomachus has mentioned only ten types in his book. | ||
| + | |style="text-align:right;"|ומיני המתיחסים רבים זכר מהם ניקומכוש בספרו עשרה מינים לבד | ||
| + | |- | ||
| + | |A sect of the ancients endeavored in the investigation of the additional to them and found ten others, so there were twenty types. | ||
| + | |style="text-align:right;"|ויחתרו כת מן הקדמונים בדרישת מה שנוסף עליהם ומצאו עשרה אחרים והיו בהם מיני היחס עשרים מינים | ||
| + | |- | ||
| + | |Yet, the most beneficial among them in the sciences are mostly only three, which are the arithmetic, the geometric, and the harmonic proportions. | ||
| + | |style="text-align:right;"|והמועילים בהם בחכמות על הרוב שלשה לבד והם {{#annot:term|2098,1769|kiqz}}המתיחסים המספריי'{{#annotend:kiqz}} ו{{#annot:term|2097,1770|ix2l}}התשברתיים{{#annotend:ix2l}} והחבוריים | ||
| + | |- | ||
| + | |First we will deal with the mentioning of these, then the mentioning of the others among the first ten types will follow. | ||
| + | |style="text-align:right;"|ונתעסק בזכרון אלו ראשונה אח"כ ימשך להם זכרון הנשארים מן העשרה מינים הראשונים | ||
| + | |- | ||
| + | |As for the ten additional, to which the ancients strived, we have no business in mentioning them because they are hardly found. | ||
| + | |style="text-align:right;"|והעשרה הנוספים אשר טרחו בהם הקדמונים אין לנו עסק בזכרונם הנה למעוט מציאותם | ||
| + | |- | ||
| + | | | ||
| + | *The arithmetic proportion: the proportions that fall between three or more terms, succeeding by an equal excess between them. | ||
| + | |style="text-align:right;"|ואולם {{#annot:term|2098,1769|zjqy}}המתיחסים המספריים הם המתיחסים{{#annotend:zjqy}} הנופלים בין גבולים אם שלשה או יותר מזה נמשכים על שווי מה שביניהם מהתוספת והיתרון | ||
| + | |- | ||
| + | | | ||
| + | ::Such as: | ||
| + | ::*<math>\scriptstyle{\color{blue}{2;\;3;\;4}}</math> succeeding one by one | ||
| + | |style="text-align:right;"|כשנים והשלשה והארבעה המוסיפים אחד אחד | ||
| + | |- | ||
| + | | | ||
| + | ::*<math>\scriptstyle{\color{blue}{5;\;7;\;9}}</math> succeeding two by two | ||
| + | |style="text-align:right;"|וכחמשה והשבעה והתשעה המוסיפים שנים שנים | ||
| + | |- | ||
| + | | | ||
| + | :But, the ratio between these numbers is not one in quantity, i.e. the excess [?]: | ||
| + | |style="text-align:right;"|ואין היחס בין אלו המספרים אחד בכמות רצוני היתרון | ||
| + | |- | ||
| + | | | ||
| + | ::*<math>\scriptstyle{\color{blue}{4:3}}</math> sesquitertian ratio | ||
| + | |style="text-align:right;"|כי יחס הארבעה אל השלשה יחס הדמיון המוסיף שליש | ||
| + | |- | ||
| + | | | ||
| + | ::*<math>\scriptstyle{\color{blue}{3:2}}</math> sesquialter ratio | ||
| + | |style="text-align:right;"|ויחס השלשה אל השנים יחס הדמיון המוסיף חצי | ||
| + | |- | ||
| + | | | ||
| + | ::*<math>\scriptstyle{\color{blue}{9:7}}</math> superbiseptian ratio | ||
| + | |style="text-align:right;"|ויחס התשעה אל השבעה יחס דמיון המוסיף שתי שביעיות | ||
| + | |- | ||
| + | | | ||
| + | ::*<math>\scriptstyle{\color{blue}{7:5}}</math> superbiquintan ratio | ||
| + | |style="text-align:right;"|ויחס השבעה אל החמשה יחס הדמיון המוסיף שתי חמישיות | ||
| + | |- | ||
| + | | | ||
| + | ::Also for the discontinuous: | ||
| + | |style="text-align:right;"|וכן יהיה ג"כ בנבדלים | ||
| + | |- | ||
| + | | | ||
| + | ::Such as: <math>\scriptstyle{\color{blue}{2;\;5}}</math> and <math>\scriptstyle{\color{blue}{4;\;7}}</math> and <math>\scriptstyle{\color{blue}{8;\;11}}</math> | ||
| + | |style="text-align:right;"|כמו השנים והחמשה והארבעה והשבעה והשמנה והאחד עשר | ||
| + | |- | ||
| + | | | ||
| + | ::For, the ratio between these six numbers is an arithmetic ratio from the aspect of the difference, and the terms exceed by one quantity, which is three. | ||
| + | |style="text-align:right;"|כי היחס אשר בין אלו המספרים הששה יחס מספריי על צד ההבדל והגבולים בו מוסיפים בכמות אחת והוא השלשה | ||
| + | |- | ||
| + | | | ||
| + | ::Yet the proportion is not one: | ||
| + | |style="text-align:right;"|ואולם ההתיחסות אינו אחד | ||
| + | |- | ||
| + | | | ||
| + | ::*<math>\scriptstyle{\color{blue}{11:8}}</math> is the same and a quarter and an eighth. | ||
| + | |style="text-align:right;"|לפי שיחס האחד עשר אל השמנה יחס הדמיון המוסיף רביעית ושמינית | ||
| + | |- | ||
| + | | | ||
| + | ::*<math>\scriptstyle{\color{blue}{7:4}}</math> is the same and a half and a quarter. | ||
| + | |style="text-align:right;"|ויחס השבעה אל הארבעה יחס הדמיון המוסיף חצי ורביעית | ||
| + | |- | ||
| + | | | ||
| + | ::*<math>\scriptstyle{\color{blue}{5:2}}</math> double sesquialter ratio | ||
| + | |style="text-align:right;"|ויחס החמשה אל השנים יחס הכפל המוסיף חצי | ||
| + | |- | ||
| + | | | ||
| + | :*Among the properties of this proportion: the sum of the extremes, of the continuous, is double the mean <math>\scriptstyle a_1+a_3=2a_2</math>; and of the discontinuous, it is equal to the sum of the means <math>\scriptstyle a_1+a_4=a_2+a_3</math>. | ||
| + | |style="text-align:right;"|ומסגלות אלו המתיחסים וזה האמצעיות שמקובץ הקצוות במתדבקים מהם כפל האמצעי ובנבדלים שוה למקובץ האמצעיים | ||
| + | |- | ||
| + | | | ||
| + | :*Also, among their properties: the ratio between the two smaller terms, of the continuous and the discontinuous, is always greater than the ratio between the greater terms. | ||
| + | ::<math>\scriptstyle a_2:a_1>a_3:a_2</math> and <math>\scriptstyle a_2:a_1>a_4:a_3</math> | ||
| + | |style="text-align:right;"|ומסגלותם גם כן שהיחס שבין שני הגבולים הקטנים במדובקים מהם והנבדלים יותר גדול תמיד מהיחס אשר בין שני גבולים הגדולים | ||
| + | |- | ||
| + | | | ||
| + | :*Among them: the product of one of the extremes by the other is always smaller than the square of the mean by the square of the excess, for the continuous <math>\scriptstyle a_2^2-\left(a_1\sdot a_3\right)=\left(a_2-a_1\right)^2</math>. | ||
| + | |style="text-align:right;"|ומהם שהכאת אחת הקצוות באחרת יותר מעט תמיד ממרובע האמצעי במדובקים כמו מרבע התוספת | ||
| + | |- | ||
| + | | | ||
| + | :*From these properties, and from the knowledge of the properties of the numbers that preceded them, the mean of this proportion is known by the two extremes: | ||
| + | |style="text-align:right;"|ומאלו הסגלות וממה שקדם להם מהידיעה בסגלות המספרים יודע האמצעי באלו המתיחסים מצד שתי הקצוות | ||
| + | |- | ||
| + | | | ||
| + | ::*For the continuous: by taking half their sum and the result is the mean. <math>\scriptstyle a_2=\frac{1}{2}\sdot\left(a_1+a_3\right)</math>. | ||
| + | |style="text-align:right;"|וזה במתדבקים כאשר ילקח חצי מקבצם ומה שהיה הוא האמצעי | ||
| + | |- | ||
| + | | | ||
| + | :::Since every number is equal to half the sum of its two sides, as is known. <math>\scriptstyle n=\frac{1}{2}\sdot\left[\left(n-1\right)+\left(n+1\right)\right]</math>. | ||
| + | |style="text-align:right;"|לפי שכל מספר שוה לחצי מקובץ שתי {{#annot:term|1465,327|uyxT}}פאותיו {{#annotend:uyxT}}כמו שידעת | ||
| + | |- | ||
| + | | | ||
| + | ::*For the discontinuous: the two means are not defined by knowing the two extremes alone - many means can be found between them. | ||
| + | |style="text-align:right;"|ואמנם בנבדלים הנה לו יוגבלו שני האמצעיים מהידיעה בשתי הקצוות לבד אבל כבר אפשר שימצאו ביניהם אמצעיים רבים | ||
| + | |- | ||
| + | | | ||
| + | :::If the two means are unknown together, but their sum is known, or the difference between them is known, or the ratio of one of them to the other is known, then finding each one of them by its number is possible. | ||
| + | |style="text-align:right;"|ואם יהיו האמצעיי' נעלמים יחד והיה מקובצם ידוע או מותר מה שביניהם ידוע ויחס אחד מהם אל האחר ידוע הנה אפשר מציאות כל אחד מהם על מנינו | ||
| + | |- | ||
| + | | | ||
| + | :::Yet, if none of these things is known, the way of extracting and finding each of them cannot be mentioned here. | ||
| + | |style="text-align:right;"|ואם לא יהיה דבר מאלו הדברים ידוע יהיה דרך הוצאתם ומציאות כל אחד מהם ממה שאין דרך לזכרו הנה | ||
| + | |- | ||
| + | | | ||
| + | *The geometric proportion: the ratio between the terms is one, but the differences differ as the diversity of the numbers. | ||
| + | |style="text-align:right;"|ואולם {{#annot:term|2097,1770|Fvij}}המתיחסי' התשברתיים{{#annotend:Fvij}} הם אשר יהיה היחס אשר בין גבוליהם אחד והמותרות מתחלפות בחלוף המספריים | ||
| + | |- | ||
| + | | | ||
| + | ::Such as: | ||
| + | ::*<math>\scriptstyle{\color{blue}{1;\;2;\;4}}</math> for the continuous. | ||
| + | |style="text-align:right;"|כמו האחד והשנים והארבעה במתדבקי' | ||
| + | |- | ||
| + | | | ||
| + | ::*<math>\scriptstyle{\color{blue}{1;\;2;\;3;\;6}}</math> for the discontinuous. | ||
| + | |style="text-align:right;"|והאחד והשנים והשלשה והששה בנבדלים | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{4:2=2:1}}</math> | ||
| + | |style="text-align:right;"|וזה שיחס ד' ב' כיחס ב' א' | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{4-2>2-1}}</math> | ||
| + | |style="text-align:right;"|והמותר בין ד' ב' יותר ממותר שבין ב' א' | ||
| + | |- | ||
| + | | | ||
| + | :::Also for: <math>\scriptstyle{\color{blue}{6;\;3}}</math> and <math>\scriptstyle{\color{blue}{2;\;1}}</math> whose ratio is the same, but their differences are different. | ||
| + | |style="text-align:right;"|וכן בו' ג' וב' א' שיחסם אחד ומותרם מתחלפים | ||
| + | |- | ||
| + | | | ||
| + | :*Among the properties of this proportion: product of one of the extremes by the other is always equal to the square of the mean, of the continuous <math>\scriptstyle a_1\sdot a_3=a_2^2</math>; and it is equal to the product of one of the means by the other, of the discontinuous <math>\scriptstyle a_1\sdot a_4=a_2\sdot a_3</math>. | ||
| + | |style="text-align:right;"|ומסגלות אלו המתיחסים וזה האמצעיות שהכאת אחת הקצוות תמיד באחרת שוה למרבע האמצעי במתדבקי' ולהכאת אחד האמצעיים באחר בנבדלים | ||
| + | |- | ||
| + | | | ||
| + | ::Therefore, the finding and the extraction of the mean, for the continuous, is by taking the root of the product of one of the extremes by the other and the result is the mean. <math>\scriptstyle a_2=\sqrt{a_1\sdot a_3}</math> | ||
| + | |style="text-align:right;"|ולזה היה מציאות האמצעי והוצאתו אם במתדבקים הוא כאשר ילקח שרש הכאת אחת הקצוות באחרת ומה שהיה הוא האמצעי | ||
| + | |- | ||
| + | | | ||
| + | ::For the discontinuous, one of the two means should be known, together with each of the extremes, in order to know the other mean by this way, and this is by the division of the product of one of the extremes by the other, by [the known mean] <math>\scriptstyle a_2=\frac{a_1\sdot a_4}{a_3}</math>. | ||
| + | |style="text-align:right;"|ואם בנבדלים הנה יצטרך עם הידיעה בכל אחת מהקצוות שיהיה אחד משני האמצעיים ידוע כדי שנדע מזה הצד האמצעי האחר וזה בחלוק הכאת אחת הקצוות באחרת עליו | ||
| + | |- | ||
| + | | | ||
| + | ::If both [two means] are unknown together, while their sum is known, or the difference between them is known, or the ratio of one of them to the other is known, then finding each of them by its number is possible. | ||
| + | |style="text-align:right;"|ואם היו מוסכלים יחד יהיה מקובצם ידוע או מותר מה שביניהם ידוע או יחס אחד מהם אל האחר ידוע הנה אפשר מציאות כל אחד משניהם על מנינו | ||
| + | |- | ||
| + | | | ||
| + | ::Yet, if none of these things is known, the way of extracting and finding each of them is not described here. | ||
| + | |style="text-align:right;"|ואם לא יהיה אחד מאלו הדברים ידוע יהיה דרך הוצאותם ומציאות כל אחד מהם ממה שאין דרכו שיחובר הנה | ||
| + | |- | ||
| + | | | ||
| + | *The harmonic proportion: the ratio of the greater term to the smaller is as the ratio of the difference between the greater and the mean to the difference between the mean and the smaller. | ||
| + | :<math>\scriptstyle a_3:a_1=\left(a_3-a_2\right):\left(a_2-a_1\right)</math> | ||
| + | |style="text-align:right;"|ואולם {{#annot:term|2095,1771|T8Wb}}ההתיחסות החבוריי{{#annotend:T8Wb}} הוא אשר יהיה בו יחס גדול שבגבוליו אל הקטן כיחס מותר מה שבין הגדול והאמצעי אל מותר מה שבין האמצעי והקטן | ||
| + | |- | ||
| + | | | ||
| + | ::Such as: <math>\scriptstyle{\color{blue}{3;\;4;\;6}}</math>. | ||
| + | |style="text-align:right;"|כמו השלשה והארבעה והששה | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{a_3:a_1=6:3=2:1=\left(6-4\right):\left(4-3\right)=\left(a_3-a_2\right):\left(a_2-a_1\right)}}</math> | ||
| + | |style="text-align:right;"|כי יחס הששה אל השלשה כיחס מותר מה שבין הששה והארבעה והוא שנים אל מותר מה שבין הארבעה והשלשה והוא אחד | ||
| + | |- | ||
| + | | | ||
| + | :The need of this type of proportion is concerns with the science of composing the melodies that is called music, since the ratio of the intervals determined by it is found in this type alone, as will be stated below, therefore it is called harmonic. | ||
| + | |style="text-align:right;"|והצורך אל זה המין ממיני המתיחסים נוגע בחכמת חבור הלחנים הנקרא המוסיקי לפי שיחס המרחקים המסכימים בו אמנם ימצאו בזה המין לבד כפי מה שתאמר עליו אחרי זה ולכן נקרא חבוריי | ||
| + | |- | ||
| + | | | ||
| + | :The extraction of the mean term in this proportion: multiplying the difference between the extremes by the smaller term, then dividing the product by the sum of the two extremes, adding the quotient to the smaller term and the result is the mean term. | ||
| + | :<math>\scriptstyle a_2=\frac{a_1\sdot\left(a_3-a_1\right)}{a_1+a_3}+a_1</math> | ||
| + | |style="text-align:right;"|והוצאת הגבול האמצעי מגבולי ההתיחסות הזה הוא כשנכה לעולם מותר מה שבין הקצוות בקטן שבגבולים ונחלק מה שהתקבץ על מקובץ שתי הקצוות ונוסיף מה שיצא על גבול הקטן ומה שהתקבץ הנה הוא הגבול האמצעי | ||
| + | |- | ||
| + | | | ||
| + | ::For example: if 3 and 6 are known. | ||
| + | |style="text-align:right;"|דמיון זה אם נדע השלשה והששה | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{a_2=\frac{a_1\sdot\left(a_3-a_1\right)}{a_1+a_3}+a_1=\frac{3\sdot\left(6-3\right)}{3+6}+3=\frac{3\sdot3}{9}+3=\frac{9}{9}+3=1+3=4}}</math> | ||
| + | |style="text-align:right;"|הנה המותר שלשה נכה שלשה בשלשה יהיה תשעה ומקובץ הקצוות ג"כ תשעה וכשנחלק תשעה על תשעה יעלה אחד לבד נוסיף האחד על השלשה שהוא גבול הקטן ויהיה ארבעה והוא האמצעי | ||
| + | |- | ||
| + | |These three types of proportions are used in the sciences, and the received is of benefit in them. | ||
| + | |style="text-align:right;"|הנה אלו השלשה ממיני המתיחסים הם הנעשי' בחכמות והמקובל תועלת בהם | ||
| + | |- | ||
| + | | | ||
| + | *The fourth proportion: the ratio of the greater to the smaller is as the ratio of the difference between the two smaller terms to the difference between the two greater terms. | ||
| + | :<math>\scriptstyle a_3:a_1=\left(a_2-a_1\right):\left(a_3-a_2\right)</math> | ||
| + | |style="text-align:right;"|ואולם המין הרביעי הוא אשר יהיה בו יחס הגדול אל הקטן כיחס מותר מה שבין שני הגבולים הקטנים אל מותר מה שבין שני הגבולים הגדולים | ||
| + | |- | ||
| + | | | ||
| + | ::Such as: <math>\scriptstyle{\color{blue}{3;\;5;\;6}}</math>. | ||
| + | |style="text-align:right;"|כמו השלשה והחמשה והששה | ||
| + | |- | ||
| + | | | ||
| + | :This type is subcontrary to the harmonic type. | ||
| + | |style="text-align:right;"|וזה המין מקביל למין החבוריי | ||
| + | |- | ||
| + | | | ||
| + | :Its property: the product of the greater by the mean is double the product of the mean by the smaller <math>\scriptstyle a_3\sdot a_2=2\sdot\left(a_2\sdot a_1\right)</math>[incorrect for the general case] | ||
| + | |style="text-align:right;"|ויסגלהו שמוכה הגדול באמצעי כפל מוכה האמצעי בקטן | ||
| + | |- | ||
| + | | | ||
| + | ::As here: <math>\scriptstyle{\color{blue}{a_3\sdot a_2=6\sdot5=30=2\sdot15=2\sdot\left(5\sdot3\right)=2\sdot\left(a_2\sdot a_1\right)}}</math> | ||
| + | |style="text-align:right;"|כמו שיהיה בכאן מוכה ששה בחמשה והוא שלשים כפל מוכה שלשה בחמשה והוא חמשה עשר | ||
| + | |- | ||
| + | | | ||
| + | *The fifth proportion: the ratio of the mean to the smaller is as the ratio of the difference between the two of them to the difference between the mean and the greater. | ||
| + | :<math>\scriptstyle a_2:a_1=\left(a_2-a_1\right):\left(a_3-a_2\right)</math> | ||
| + | |style="text-align:right;"|ואולם המין החמישי הוא אשר יהיה בו יחס האמצעי אל הקטן כיחס מותר מה שבין שניהם אל מותר מה שבין האמצעי והגדול | ||
| + | |- | ||
| + | | | ||
| + | ::Such as: <math>\scriptstyle{\color{blue}{2;\;4;\;5}}</math>. | ||
| + | |style="text-align:right;"|כמו השנים והארבעה והחמשה | ||
| + | |- | ||
| + | | | ||
| + | :Its property: the product of the greater by the mean is double its product by the smaller <math>\scriptstyle a_3\sdot a_2=2\sdot\left(a_3\sdot a_1\right)</math> [incorrect for the general case] | ||
| + | |style="text-align:right;"|ויסגלהו שמוכה הגדול באמצעי כפל הכאתו בקטן | ||
| + | |- | ||
| + | | | ||
| + | ::Such as: <math>\scriptstyle{\color{blue}{a_3\sdot a_2=5\sdot4=20=2\sdot10=2\sdot\left(5\sdot2\right)=2\sdot\left(a_3\sdot a_1\right)}}</math> | ||
| + | |style="text-align:right;"|כמו שהכאת החמשה בארבעה והוא עשרים כפל הכאת חמשה בשנים אשר הוא עשרה | ||
| + | |- | ||
| + | | | ||
| + | *The sixth proportion: the ratio of the greater to the mean is as the ratio of the difference between the mean and the smaller to the difference between the mean and the greater. | ||
| + | :<math>\scriptstyle a_3:a_2=\left(a_2-a_1\right):\left(a_3-a_2\right)</math> | ||
| + | |style="text-align:right;"|ואולם המין הששי הנה הוא אשר יהיה בו יחס הגדול אל האמצעי כיחס מותר מה שבין האמצעי והקטן אל מותר מה שבין האמצעי והגדול | ||
| + | |- | ||
| + | | | ||
| + | ::Such as: <math>\scriptstyle{\color{blue}{1;\;4;\;6}}</math>. | ||
| + | |style="text-align:right;"|כמו האחד והארבעה והששה | ||
| + | |- | ||
| + | | | ||
| + | *The seventh proportion: the ratio of the greater to the smaller is as the ratio of the difference between both of them to the difference between the mean and the smaller. | ||
| + | :<math>\scriptstyle a_3:a_1=\left(a_3-a_1\right):\left(a_2-a_1\right)</math> | ||
| + | |style="text-align:right;"|ואולם המין השביעי הנה הוא אשר יהיה בו יחס הגדול אל הקטן כיחס מותר מה שבין שניהם אל מותר מה שבין האמצעי והקטן | ||
| + | |- | ||
| + | | | ||
| + | ::Such as: <math>\scriptstyle{\color{blue}{6;\;8;\;9}}</math>. | ||
| + | |style="text-align:right;"|כמו הששה והשמנה והתשעה | ||
| + | |- | ||
| + | | | ||
| + | *The eighth proportion: the ratio of the greater to the smaller is as the ratio of the difference between the two extremes to the difference between the greater and the mean. | ||
| + | :<math>\scriptstyle a_3:a_1=\left(a_3-a_1\right):\left(a_3-a_2\right)</math> | ||
| + | |style="text-align:right;"|ואולם המין השמיני הנה הוא אשר יהיה יחס הגדול אל הקטן כיחס מותר מה שבין הקצוות אל מותר מה שבין הגדול והאמצעי | ||
| + | |- | ||
| + | | | ||
| + | ::Such as: <math>\scriptstyle{\color{blue}{6;\;7;\;9}}</math>. | ||
| + | |style="text-align:right;"|כמו הששה והשבעה והתשעה | ||
| + | |- | ||
| + | | | ||
| + | *The ninth proportion: the ratio of the mean to the smaller is as the ratio of the difference between the two extremes to the difference between the mean and the smaller. | ||
| + | :<math>\scriptstyle a_2:a_1=\left(a_3-a_1\right):\left(a_2-a_1\right)</math> | ||
| + | |style="text-align:right;"|ואולם המין התשיעי הנה הוא אשר יהיה בו יחס האמצעי אל הקטן כיחס מותר מה שבין הקצוות אל מותר מה שבין האמצעי והקטן | ||
| + | |- | ||
| + | | | ||
| + | ::Such as: <math>\scriptstyle{\color{blue}{4;\;6;\;7}}</math>. | ||
| + | |style="text-align:right;"|כמו הארבעה והששה והשבעה | ||
| + | |- | ||
| + | | | ||
| + | *The tenth proportion: the ratio of the mean to the smaller is as the ratio of the difference between the two extremes to the difference between the mean and the greater. | ||
| + | :<math>\scriptstyle a_2:a_1=\left(a_3-a_1\right):\left(a_3-a_2\right)</math> | ||
| + | |style="text-align:right;"|ואולם המין העשירי הנה הוא אשר יהיה בו יחס האמצעי אל הקטן כיחס מותר מה שבין שני הקצוות אל מותר מה שבין האמצעי והגדול | ||
| + | |- | ||
| + | | | ||
| + | ::Such as: <math>\scriptstyle{\color{blue}{3;\;5;\;8}}</math>. | ||
| + | |style="text-align:right;"|כמו השלשה והחמשה והשמנה | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|הנה אלו הם המינים העשרה ממיני המתיחסים שיספיק לנו זכרונם כי אין לאחד מהם מבא בחכמה זולתי מה שזכרנום לפנים ואולם הביאונום על צד שלמות החלוקה והמשכתה וספירת מה שיגיעו אליו צדי ההתיחסות נשלם זה והתהלה לאל ית' שמו | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | == Music == | ||
| + | |||
| + | | | ||
|- | |- | ||
| | | | ||
| Line 4,132: | Line 6,017: | ||
== Appendix: Bibliography == | == Appendix: Bibliography == | ||
| − | Abū al- | + | Abū al-Ṣalt Umayya b. ‘Abd al-‘Azīz al-Andalusī (b. Andalusia ca. 1068 – d. Tunisia ca. 1134)<br> |
– Hebrew translation –<br> | – Hebrew translation –<br> | ||
Don Benveniste b. Lavi<br> | Don Benveniste b. Lavi<br> | ||
| Line 4,141: | Line 6,026: | ||
:1) Oxford, Bodleian Library MS Heb. d. 3/4 (IMHM: f 22729), ff. 47v-59r (cat. Neub. 2774, 4) (16th century) | :1) Oxford, Bodleian Library MS Heb. d. 3/4 (IMHM: f 22729), ff. 47v-59r (cat. Neub. 2774, 4) (16th century) | ||
:2) St. Petersburg, Inst. of Oriental Studies of the Russian Academy B 103/1 (IMHM: f 44256), ff. 1r-21v (15th century) | :2) St. Petersburg, Inst. of Oriental Studies of the Russian Academy B 103/1 (IMHM: f 44256), ff. 1r-21v (15th century) | ||
| + | :[https://www.nli.org.il/he/discover/manuscripts/hebrew-manuscripts/viewerpage?vid=MANUSCRIPTS&docid=PNX_MANUSCRIPTS990001761980205171-1#$FL56529553 St.Petersburg103] | ||
| + | <span style=color:blue>The transcript is based mainly on manuscript St. Petersburg</span> | ||
'''Bibliography:'''<br> | '''Bibliography:'''<br> | ||
*Langermann, Y. Tzvi. 2001. Studies in Medieval Hebrew Pythagoreanism: Translations and Notes to Nicomachus; Arithmological Texts, Micrologus IX, pp. 219–36. | *Langermann, Y. Tzvi. 2001. Studies in Medieval Hebrew Pythagoreanism: Translations and Notes to Nicomachus; Arithmological Texts, Micrologus IX, pp. 219–36. | ||
*Lévy, Tony. 1996. L’histoire des nombres amiables: le témoignage des textes hébreux médiévaux, Arabic Sciences and Philosophy 6, pp. 63–87. | *Lévy, Tony. 1996. L’histoire des nombres amiables: le témoignage des textes hébreux médiévaux, Arabic Sciences and Philosophy 6, pp. 63–87. | ||
Latest revision as of 07:05, 18 November 2022
Contents
- 1 Book One
- 2 Book Two
- 3 Music
- 4 Notes
- 5 Appendix: Bibliography
| The Book of Nicomachus on the Numerical Properties | ספר ניקומאכוש בסגלות המספריות |
| Don Benveniste b. Lavi, may his soul rest in heaven, translated it from Arabic to the holy language in the year 195 of creation. | העתיקו בסרגוסא דארגו' השר דון בנבשת בן לביא נ"ע מלשון הגרי ללשון הקדש שנת קצ"ה ליצירה |
| In any place where A"B is written in the work, it means: Benveniste said. | ובכל מקום הכתוב בגיליון א"ב ר"ל אמר בן בנשתי' |
Book One |
|
Introduction - Basic Definitions |
|
|
חכמת המספר חכמה עיונית נושאה המספר המשולח אליו ומיניו והכונה בה העמידה על משיגיו ועל המתחייב אליו וסגלותיו ובכלל מקריו העצמיים |
|
ומהות המספר הנה תאמר המספר קבוץ אחדים וגדרו שהוא כמות מתחלק מתקיים מאחדים |
|
ויש לוקחין האחדותיות תמורת האחדים לפי שהוא יותר מורה על טבע המספר, לפי שקבוץ האחדים אינו המספר עצמו המכוון הנה תאמו וגדרו ובכלל הודעת מהותו אבל הנספרים אשר הם נושאי המספר כקבוץ מהאנשים או מהסוסי' או זולת זה |
|
וקבוץ האחדותיות הוא המספר עצמו המקובץ מהכפלת האחדות אשר בה יאמ' לכל דבר מהנספרים אחד |
|
והמספר המשולח יתחלק ראשנה לשני מינים זוג ונפרד |
|
והזוג הוא כל מספר שיתחלק לשני חלקים שוים כשנים וארבע |
|
והנפרד הוא כל מספר שא"א שיחלק לשני חלקים שוים |
| Our intention is to add the known way of arithmetic to the mathematical science that we have prefaced and what is customary to bring concerning it according to the way that was spread from the Book of Elements that presented many principles in arithmetic, and establishing this way by the realization of this principles. | כונתינו שנחבר אל מה שהקדמנו מהחכמות הלמודיות האופן הידוע בארתימטיקי ומה שפשט המנהג להביאו בו כפי האופן אשר פשט על ספר היסודות כבר נתן שרשים רבים בחכמת המספר והנחת זה האופן אצל ההגעה באותם השרשים |
| Many of the geometric shapes that have relation with the multiplication, division and ratio, may be related to the number and the rules of number of this book may be established upon them. | וכבר אפשר שתתחברנה הרבה מהתמונות התשברתיות אשר להם התלות בהכאה והחלוק ובענייני היחס אל המספר ושתישבו ממנו משפטי המספר זה הספר והנה זה אליך |
| As for the essence of the number - some of it is already known in the Book of Categories and a hint about it was alluded in the Book of Elements, also in metaphysics one reaches to truths about it. | אמנם מהות המספר הנה כבר ידעת בספר קאטיגוריאש ממנו דבר מה ונרמז לך בספר היסודות אליו רמז עוד יבא אליך בחכמה העליונה ממנו אמתות |
| Likewise for its two parts, which are the even number and the odd number. | וכמו כן הענין בשני חלקיו אשר הם הזוג והנפרד |
| You already know the absolute prime, the absolute composite, and the relative composite from the Book of Element. | וכבר ידעת מספר היסודות הראשון והמורכב מוחלטים והמורכב בצרוף |
| You know the even and the odd, the even-times-odd, the even-times-even, and the even-times-even-times-odd, as well as the deficient number, the perfect number and the superabundant number. | וידעת הזוג והנפרד וזוג הנפרד וזוג הזוג וזוג הזוג והנפרד וידעת המספר החסר והשלם והנוסף |
| We are not obligated to simplify [?] the mentioning of these matters for you, but we will endeavor to present the properties to you. | ואיננו מחוייב אלינו הפשת זכרון אלה העניינים לך אבל שנשתדל להביא הסגלות אליך |
Properties of the Absolute Number |
|
| Mentioning the properties of the absolute number: | ונזכור סגלות המספר המשולח |
|
והנה ראשנה שבהם והיותר מפורסמת שכל מספר הנה הוא חצי שתי פאותיו והם שני המספרים הנלוים אליו מצד המעוט והרבוי במרחק שוה |
|
המשל בזה החמשה הנה הוא חצי ששה וארבעה וחצי שבעה ושלשה וחצי שמנה ושנים וחצי תשעה ואחד |
|
והנה יהיה כפלו שוה לשתי פאותיו |
|
וחציו לרביעית שתי פאותיו |
|
וכל מספר יהיה מרובעו שוה להכאת שתי פאותיו הקרובות אחת מהם באחרת עם תוספת אחד |
|
כמו מרובע שנים אשר הוא מהכאת שלשה באחד ותוספת אחד |
|
|
וכמו מרובע שלשה אשר הוא מהכאת ארבעה בשנים ותוספת אחד |
|
|
וכמו מרובע ארבעה אשר הוא מהכאת ג' בחמשה ותוספת אחד |
|
וגם נאמר שכל מספר הנה מרובעו יוסיף על מושטח שתי פאותיו הרחוקות מרחק שוה תהיינה מה שתהיינה אחת מהם באחרת כמרובע מספר המדרגות אשר ביניהם |
| ? | והנה אם תהיינה השתי פאות הקרובות והנה המדרגה היא הראשונה יוסיף כמרובע שלשה |
|
וכל מספר הנה מרחקו במדרגות מכפלו אם כשתקח אותו ראשון למדרגות הנה הוא כמו מספרו ותוספת אחד |
|
ואם כשתקח ראשית המדרגות המספר הנלוה אחריו הנה הוא כמספר מה שבו מן האחדים |
|
המשל בזה שבין ארבעה ושמנה |
|
לפעמים ד' ה' ו' ז' ח' והנה זה חמשה |
|
ולפעמים ה' ו' ז' ח' וזה כמו מספר מה שבו מן האחדים |
|
וכל מספר מרחקו משלשה כפליו הנה הוא בכמו שיעור אחדיו מוכים בשנים |
|
אם בתוספת אחד |
|
אם בזולת תוספת אחד כפי מה שידעת אותו |
|
כמו השנים אשר מרחקם מו' שהוא ג' דמיוניו הוא כמספר הכאתו בשנים בתוספת ובזולת תוספת |
|
וכל מספר מרחקו מד' כפליו הוא כהכאתו בג' מהמספר בתוספת |
|
ובזולת תוספת |
|
|
ובכלל הנה המרחק בכל מקום הוא שנגרע מקריאת הכפלים אחד ונכה המספר במה שנשאר ואח"כ נוסיף או לא נוסיף |
|
|
וכל מספר הנה מרחקו במרובעו בשעור הכאתו במספר אשר לפניו בתוספת אחד או בזולת תוספת |
|
כמו הכאת השנים באחד אשר הוא מרחקו ממרובעו כשלא נוסיף |
|
|
והכאת השלשה בשנים אשר הוא מרחק השלשה ממרובעו בשלא נוסיף |
|
וכמו כן כל מספר הנה מרובעו שוה להכאתו בתוספת אחד במספר אשר לפניו ותוספת אחד |
|
כמו מרובע השנים אשר הוא שוה להכאת השלשה באחד ותוספת אחד |
|
|
ומרובע השלשה אשר הוא שוה להכאת הארבעה בשנים ותוספת אחד |
|
וכל מספר הנה מרחקו מהכאתו במספר אשר לפניו הוא כמו מרבע המספר אשר לפניו כשלא יתוסף |
|
המשל בזה שמרחק השלשה מהכאתו בשלשה הוא כמו מספר מרבע השנים |
|
|
ומרחק הארבעה מהכאתו בשלשה הוא כמו מספר מרבע שלשה רצוני בזה כשלא יתוסף |
|
וכל מספר הנה מרחקו מהכאתו במספר אשר אחריו כמו מספר מרובעו |
|
וכל מספר הנה מרחקו ממעוקבו הוא כמו הנשאר ממעקבו אחר שיהיה בגרע ממנו |
|
כי בין השנים ומעקבו ששה וכמו בין מאלו והלאה עד אין תכלית |
|
וכל מספר הנה בינו ובין מעקבו מהמדרגות כמו הכאתו באשר ימשך אליו אחר כן הכאת זה כלו באשר לפניו |
|
כמו שנים בג' אחר כן באחד |
|
|
ושלשה בארבעה אחר כן בשנים |
|
|
וכל מספר הנה בינו ובין העולה מהכאתו הנקרא בערבי מאל מאלה כמו הכאת מרבעו מחובר אל המספר הנמשך לזה המספר במה שעלה מהכאתו עם המספר אשר לפניו |
|
כמו שבין מאל מאלה של שנים ושנים הוא י"ד ויתחדש מהכאת מרבע שנים מחובר עם שלשה שהוא ז' בהכאת שנים באחד |
| וכמו כן מה שימשך והנה מה שנתקבץ בזה ונשוב אל בחינת סגלות המספרים הנמשכים | |
|
|
וכל מספר מרבעו כאשר נכפל ונוסף עליו שנים הנה הוא שוה למקובץ שני מרובעי פאותיו הקרובות |
|
המשל בזה פי' הכאת עשרה בעצמו בתוספת שנים והוא מאתים ושנים הנה הוא שוה להכאת תשעה בעצמו והוא שמנים ואחד ולהכאת אחד עשר בעצמו והוא מאה ועשרים ואחד |
|
וכל מספר הנה מרובעו כאשר יכפל ויתוסף עליו שמנה הוא שוה למקובץ שני מרובעי שתי פאותיו השניות |
|
המשל בזה עשרה אשר מרובעו כשיעשה בו זה יהיה מאתים ושמנה והוא שוה להכאת שמנה בעצמו |
|
וכל מספר אשר יכפל מרובעו ויתוסף עליו שמנה עשר הנה יהיה שוה לשני מרבעי פאותיו השלישיות |
|
המשל בזה מאתים ושמנה עשר אשר הוא שוה להכאת שבעה בעצמו ושלשה עשר בעצמו |
|
ואמנם בשתי פאות הרביעיות הנה התוספת שנים ושלשי' |
|
ובשתי פאות החמישיות חמשים |
|
והסדר בזה שהתוספת הראשון הוא הכאת הזוג הראשון והוא שנים בנפרד הראשון והוא האחד |
|
והתוספת השני על זה התוספת הוא הכאת הזוג הראשון בנפרד אשר ימשך אל האחד והוא שלשה |
|
והתוספת השלישית על אלו התוספות המקובצות הוא הכאת שנים בנפרד השלישי אחר האחד |
|
|
וכמו כן כל מספר הנה מרובעו כאשר יכפל ויתוסף עליו ד' שוה לשני שטחי פאותיו היורדות ושתי פאותיו העולות כאשר יקובצו |
|
המשל בזה מאתים וארבעה אשר הוא שוה להכאת תשעה בשמנה ואחד עשר בשנים עשר |
|
|
ואמנם המושטחים הנמשכים לשני אלו מהכאת הפאה היורדת השנית ביורדת השלישית והעולה שנית בעולה שלישית הנה יוסיפו על כפל זה בשנים עשר |
|
|
ואשר ימשכו להם בעשרים וארבעה |
|
|
ואשר ימשכו להם בארבעים |
|
והסדר בזה שנכה התוספת הראשון בזוג הראשון ויהיה שמנה ותוסיפם |
|
אח"כ [ת]כה אותו במספר אשר ימשך אליו והוא שלשה ויהיה שנים עשר ותוסיף אותם |
|
אח"כ נכה אותו במספר אשר ימשך אליו והוא ארבעה והנה יהיה ששה עשר ותוסיף אותם |
|
|
וכל מספר הנה כפל מרבעו כאשר יתוסף עליו ששה הוא שוה למושטח פאתו היורדת הקרובה בפאתו היורדת השלישית ולמושטח פאתו העולה הקרובה בפאתו העולה השלישית |
|
המשל בזה מאתים וששה אשר הוא שוה להכאת תשעה בשבעה ואחד עשר בשלשה עשר |
|
|
וכאשר תכה הקרובה אשר בשני צדדיו ברביעית יהיה התוספת שמנה ולא יסורו התוספות להשתנות בשנים שנים |
|
|
[1]כל מספר הנה כפל מרובעו כאשר יתוסף עליו ששה עשר יהיה שוה למשטח הפאה השנית היורדת ברביעית היורדת והשנית העולה ברביעית העולה |
|
המשל בזה מקובץ מושטחי שמנה בששה ושנים עשר בארבעה עשריו הנה זה מאתים וששה עשר |
|
|
וכאשר תכה השניות בחמישיות יהיה התוספת עשרים |
|
|
ואם תכה אותם בששיות יהיה התוספת עשרים וארבעה וכזה ילכו בהדרגה בהעדפת ארבעה ארבעה |
|
|
ואם תהיינה השתי פאות השלישיות הכאה ראשונה בחמישיות יהיה התוספת שלשים שלשים |
|
|
וכאשר תכה אותם בששיות יהיה התוספות שלשים וששה |
|
|
ואם תכה אותם בשביעיות יהיה התוספות שנים וארבעים ולא יסורו התוספות מלכת בהדרגה ששה ששה ועל זה הסדר במה שאחר זה מהפאות |
Arithmetic Progression |
|
| ונתחיל אליך בסגלות המספרים הנמשכים המשכם הטבעי | |
| [The number of] their terms is necessarily either odd or even. | ונאמר שמדרגותיהם הם לא ימלט אם שתהיינה נפרד ואם שתהיינה זוג |
|
ואם תהיינה נפרד ימצא להם אמצעי בלי ספק |
|
וזה האמצעי יהיה תמיד חצי שתי הפיאות מקובצות |
|
ורצוני בשתי פאות שני מספרים או מספר אחד מרחקם בסדר מהאמצעי מרחק שוה אחד מהם מצד החסרון והאחר מצד התוספת |
|
כמו התשעה והאחד אשר הם שתי פאות החמשה וחמשה חצי מקובצם |
|
והוא ג"כ חצי השמנה והשנים אשר הם ג"כ שתי פאותיו |
|
וחצי השבעה והשלשה |
|
והששה והארבעה |
|
והיותר הרחוקות התשעה והאחד |
|
וכל מספר הוא אמצעי הנה הוא חצים |
|
ואם תהיינה המדרגות זוג עד שיהיו תמורת האמצעי האחד שני אמצעיים יחד |
|
הנה יהיו שני האמצעיים מקובצים כמו השתי פאות מקובצות תהיינה מה שתהיינה |
|
המשל בזה הארבעה והחמשה בין האחד והשמנה הנה הם מקובצים שוים לאחד ושמנה ולשנים ושבעה ולשלשה וששה |
It follows necessary from this rule that the [sum of] every two sides of a number is equal to the [sum of] the other corresponding [sides]. 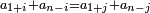
|
ויתחיב מכלל זה שתהיינה כל שתי פאות למספר מה שוות אל האחרות אשר בגילם |
Sums |
|
|
ומהמסגלות הנתלות בקבוץ בעלי המדרגות שאנחנו כאשר נוסיף על הגעת המספר האחרון המתחיל מן האחד אחד ונכה אותו בחצי מספר האחרון המדרגות יהיה העולה שוה לכלל כלם |
|
המשל בזה שיהיה ארבעה הוא האחרונה שבמדרגות |
|
|
והנה אתה כשתוסיף אחד על הארבעה ויהיו חמשה ותכה בחצי מספר המדרגות שהם ארבעה וחציו שנים יהיה העולה עשרה מקובץ מה שבין האחד עד הארבעה |
|
ואם תרצה מן האחד עד החמשה |
|
|
תוסיף על החמשה אחד ויהיו ששה בחצי מספר המדרגו' שהוא שנים וחצי ויהיה העולה חמשה עשר |
|
וגם כן הנה מקובץ כל שתי קצוות מבעלי הדרגה וסדר הן שתהיינה מן האחד או מן זולתו כאשר הוכה בחצי המדרגות או הוכה חציו בכל המדרגות הנה יהיה מה שיתקבץ כמו כלל מקובץ אותם המדרגות |
|
והנה תהיינה הראשונה שבמדרגות שנים והאחרונה ששה |
|
|
ונקבץ אותם ויהיו שמנה ונכה אותם בחצי מספר המדרגו' והוא שנים וחצי והנה הוא עשרים |
|
|
או נכה חצים במספר המדרגות על השלמות ויהיה ארבעה בחמשה וזה עשרים והוא שוה למקובץ שנים ושלשה ארבעה חמשה ששה |
|
ומהסגלות הנתלות בקבוץ שכל מספרים נמשכים ולא ימשך התוספת באחדים אבל על דרך השניות או השלישיות או זולת זה כל עוד שיהיו הולכים על הדרגה ומנהג אחד ותהיה תחילתם איך שתהיה הנה הכאת מספר המדרגות מחוסר ממנו אחד במספר אשר יפול בו ההעדף כמו השניות או השלישיות או זולת זה ממה שיעדיפו בו המדרגות מוסף עליו אשר ממנו ההתחלה הוא שוה למספר האחרון |
|
ואם יתוסף פעם אחרת והוכה במספר המדרגות כמות שהוא הנה יהיה כמו כפל כלל המקובץ |
|
המשל בזה אלו אמר לך אומר חמשה מספרים נמשכים מתחילים מן הארבעה ובין כל שני מספרים שלשה בענין שיהיה ההעדף בארבעה ארבעה מה הוא האחרון שבהם וכמה מקובצם |
|
|
הנה כשתגרע אחד מן החמשה עד שיגיע לך ארבעה ותכה אותו במספר ההעדף והוא ארבעה יהיה ששה עשר וכאשר תוסיף עליו הראשון שבהם יהיה עשרים והנה כבר יצא לך המספר האחרון |
|
לפי שמדרגות המספרי' תהיינה ארבעה אח"כ שמנה אח"כ שנים עשר אח"כ ששה עשר אח"כ עשרים |
|
|
והנה כאשר תוסיף על עשרים ארבעה ג"כ יהיה ארבעה ועשרים ואם תרצה תכה אותו בחמשה ויהיה מאה ועשרים והנה תקח חציו והוא מקובץ המדרגות |
|
ואם תרצה תכה חציו במדרגות או כלו בחצי המדרגות שיעשה הנה הוא תשובת השאלה |
|
ומהסגלות הנתלות בקבוץ שכל מספרים נמשכים מתחילים מן האחד כאשר קובצו מתחילים מן האחד עד האחרון שבהם ואחרי כן דרך חזרה מן האחרון שבהם אל האחד כמו אחד שנים שלשה ארבעה שלשה שנים אחד הנה מקובצם שוה למרובע המספר האחרון |
|
כי מקובץ מה שהמשלנו בו ששה עשר |
|
והנה יגיע זה לפי שמקובץ כפל המספרים אשר תחת הנמשכים האחרונים עם אשר במדרגה האחרונה שוה למרובע המספר האחרון |
|
ומהסגלות הנתלות בקבוץ שאתה כאשר תקבץ מספרים נמשכים מן האחד הנה המקובץ הראשון דמיון וחצי המספר האחרון והמקובץ השני כפל המספר האחרון והמקובץ השלישי כפל וחצי המספר האחרון והמקובץ הרביעי שלשה כפלי המספר האחרון והמקובץ החמישי שלשה כפלים וחצי המספר האחרון וכן לבלתי תכלית |
|
המשל בזה אחד ושנים הנה הוא דמיון וחצי השנים |
|
|
ואחד ושנים ושלשה הנה הוא כפל השלשה |
|
|
ואחד ושנים ושלשה וארבעה הנה הוא כפל וחצי הארבעה |
|
|
ואחד ושנים ושלשה וארבעה וחמשה וששה הנה הוא שלשה כפלים וחצי ששה |
|
וגם כן הנה כל המספרים הנמשכים יקובצו זה הקבוץ הנה המקובץ הראשון יהיה כמו המספר הנמשך אליו והמקובץ השני דמיון וחצי מהמספר הנמשך אליו והמקובץ השלישי כפל המספר הנמשך אליו וכמו כן אל בלתי תכלית |
|
והמשל בזה בהאחד והשנים כמו השלשה |
|
|
והאחד והשנים והשלשה כמו דמיון וחצי מן הארבעה |
|
|
וכאשר תוסיף הארבעה יהיה כפל חמשה |
|
|
וכאשר תוסיף חמשה יהיה כפל וחצי ששה וכן אל בלתי תכלית |
|
ומהסגלות הנתלות בקבוץ שאתה כאשר תקבץ נפרדים נמשכים מתחילים מן האחד ותקבץ אחריהם זוגות נמשכים מן השנים כמספרם הנה המקובץ הראשון מהזוגות יהיה דמיון וחצי המקובץ הראשון מהנפרדים והמקובץ השני דמיון ושלישיתו והמקובץ השלישי דמיון ורביעיתו ויהיה כל מקובץ יוסיף בחלק נקרא על שם מספר מדרגתו ויהיה מספרו מספר מדרגתו |
|
המשל בזה השנים והארבעה הנה יוסיף על האחד והשלשה החצי |
|
|
וכאשר תוסיף בזה ששה ובזה חמשה יהיה זה דמיון ושליש זה |
Types of Numbers |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Returning to the presentation of the properties of the first categorization of the number from the aspect of the quality of its divisibility to equals and unequals, which are the even and the odd. | ונשוב עתה אל הבאת סגלות החלוקה הראשונה מהמספר מצד איכות החלקו אל שוים ובלתי שוים והוא הזוג והנפרד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| What was discussed concerning it in the book of the Elements will also be presented. | ועוד נביא מה שדובר בו בספר היסודות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The natural successive odds and evens are subject to association acquired from their type: | וכבר יפול ביניהם שתוף נקנה מסוגם במה שימשך מהנפרדים והזוגו' המשכות טבעי המשכות מיני המספר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The terms exceed by one excess | וזה כלו שתהיינה המדרגות עודפות בהעדף אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם העדף ההמשכות הטבעי למיני המספר הנה באחד אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם העדף הנפרדים והזוגות הנמשכים בטבע הנה בשנים שנים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
לפי שכל נפרד כאשר יתוסף עליו אחד יהיה זוג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אחר כן כשיתוסף עליו אחד אחד יהיה נפרד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אח"כ כשיתוסף עליו אחד יהיה זוג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ויהיה בין הנפרד והנפרד אשר ילוה אליו שנים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ובין הזוג והזוג אשר ילוה אליו שנים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ויחויב שיהיה כל אמצעי במדרגות הנפרדים אשר על ההמשך הטבעי ומדרגות הזוגות אשר על זה ההמשך כמו חצי מקובץ איזה שתי פאות שתהיינה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
לפי שהן פאות זה האמצעי בעצמו בסדור הטבעי למספר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכל שני אמצעיים מקובצים כמו כל שתי פאות מקובצות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
לפי שבאלה השני אמצעיים תהיינה שתי פאות למספר הנופל בסדר המספרים הטבעיים ביניהם והנה יחויב שיהיה שוה מקובצם למקובץ אלה שתי הפאות האחרות כפי מה שקדם באורו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואין זה הענין נוהג בין הנפרדים הנמשכים והזוגות הנמשכי' לבד אבל בין כל המספרים העודפים על השווי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולזה תמצא זאת הסגלה ג"כ בסדר מדרגות מני הנפרד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Thus, this association should be stated before all the properties. | והנה זה ההשתתפות ראוי שנאמר אותו קודם הסגלות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Now, we will concentrate in mentioning the properties | ונתבודד עתה בזכרון הסגלות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Odd Number |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Starting with the properties of the odd number | ונתחיל בסגלות הנפרד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
The known and mentioned properties are that it does not consist of evens at all and not from an even number of odd numbers.
|
ונאמר אמנם הסגלות הידועות והנזכרות מאשר הוא לא יתרכב מזוגות כלל ולא מנפרדים במספר זוג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
There is no number of its type in it, whose remainder is of its type
|
ושלא ימצא בו מסוגו מספר ישאיר מה שאחריו מסוגו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
There is no number of its parallel type in it, whose remainder is of its parallel type
|
ושלא ימצא בו מסוג מקבילו מספר ישאיר מה שאחריו מסוג מקבילו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| What is said about whatever is applied to these properties in the book of the Elements is sufficient. | ומה שירוץ מרוצת אלו הסגלות הנה נסתפק במה שנאמר בספר היסודות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Its properties that will be discussed are the properties related to the sequence, their being successive by the way of succession | ונדבר מסגלותיו סגלות נתלות בסדור היותם נמשכים על דרך ההמשך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומסגלותיו שמקובציו על דרך ההמשך מן האחד יהיה מרובע לעולם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כמו האחד והשלשה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|
ואח"כ האחד והשלשה והחמשה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|
ואח"כ האחד והשלשה והחמשה והשבעה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומסגלותיו שכל מרבע מאלו הנה צלעו מספר המדרגות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כמו הארבעה והוא מקובץ שתי המדרגות והנה גדרו שנים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והתשעה והוא מקובץ שלשה מדרגות והנה גדרו שלשה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומסגלותיו שאתה כשתרצה לדעת הגעת מספר יפול במדרגה ידועה מן האחד כמו העשירית והאחת עשרה דרך משל וזולת זה הנה תכה מספר המדרגה ותהיה העשירית ומספרה שני עשרה בשנים ויהיו עשרים והנה תגרע מהם אחד ויהיו תשעה עשר והנה הוא מספר המדרגה העשירית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואמנם ענין האמצעי והשני אמצעיים עם השתי פאות הנה הוא ממה שידעת אותו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומסגלותיו שכל אחד מן האחדים ישוב בששי ממנו אליו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
המשל בזה שהאחד ישוב בששי והוא האחד עשר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|
ועוד בששי אחר הששי והוא האחד ועשרים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|
והשלשה ישוב לששי והוא השלשה עשר וכן על הדרך הזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומסגלותיו שכל נפרד ראשון כאשר תוציא קוו על מספרו יכלה אל מורכב ימשכו אליו ראשונים הם חסרי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כמו השלשה כי הנה השלישי ממנו והוא תשעה מורכב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והחמשה כי הנה החמישי ממנו והוא חמשה עשר מורכב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וסגלה אחרת שהראשון שבמספרים הבלתי מורכבים והוא שלשה יביא כשתוציא קוו הראשון אל נגדר אחר כן לא יביא[2] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והשני והוא החמשה יביא בהקוות השני אל נגדר אצל עשרים וחמשה אח"כ לא יבוא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועל הדרך הזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וסגלה אחרת שהרביעי אחר הנגדר הראשון והוא אחד נגדר והוא תשעה והשמיני אחר הנגדר הראשון והשנים עשר אחר הנגדר השני והששה עשר אחר הנגדר השלישי וכן בתוספת ארבעה ארבעה[3] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכל בית ומדרגה שתפול בו נגדר הנה תהיה הכאת זה הנגדר שוה לכפל מספר המדרגה מוסף עליו אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כי המספר המרובע הראשון הוא תשעה והוא במדרגה הרביעי' מן המספרים הנפרדים וכפל הארבעה שמנה והנה תוסיף עליו אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והבית השנים עשר מהשלשה יפול בו חמשה ועשרים והוא שוה לכפל שנים עשר כשתוסיף עליו אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| When the natural successive odds are set in a square table, properties are seen from the aspect of the shape. | וכאשר נניח מהנפרדים הנמשכים בטבע לוח מרובע יראו בו סגלות מצד התמונה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Also, when a triangle table is set. | וכן כאשר נניח לוח משלש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונתחיל במרובע ונשים אותו חמשה על חמשה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונאמר שכל שתי וערב ממנו יהיה קטר התמונה או לא יהיה הנה מקובץ שני קטריו הם שוים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם אשר על הקטר הנה מקובץ כל אחד מהשני קטרים אשר בזאת התמונה קכ"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם אשר אינם על הקטר הנה כמו השתי וערב אשר בשתי שורות שאחת מהן ג' ט"ו כ"ז והשנית ז' ט"ו כ"ג כי כל אחד משני אלו הקטרים ארבעים וחמשה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונמצא מקובץ שתי קצוות שורה כל שתי וערב שוה למקובץ שתי קצוות השורה האחרת | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונמצא מקובץ בתי כל מרובע בנוי מאלה המספרים על המשכם שוה למרבע מרבע מספר בתי הצלע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כי אתה כשתבנה מרבע צלעו שני בתים ויהיו מספריו כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
יהיה מקובץ זה ששה עשר והוא מרבע מרבע שנים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר תהיינה צלעותיו משלשה בתים כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עד שיהיו מספריו א' ג' ה' ז' ט' י"א י"ג ט"ו י"ז הנה יגיע מקובץ זה לשמנים ואחד והוא מרבע מרבע השלשה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונמצא הקטר בכל אלו שוה למעקב זה המספר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
המשל בזה בלוח הגדול אשר בתיו חמשה הנה קטרו קכ"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ובשני קטרו שמנה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ובשלישי קטרו כ"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועל הדרך הזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה כאשר תבנה מהם תמונה משלשת על זאת הצורה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תמצא כל המספרים היורדים מן האחד אל נפילת העמוד מרבעים על הסדר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותמצא מקובץ מה שבטור אחד ברחב מעקב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כמו מקובץ ג' וה' ומקובץ ז' ט' וי"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Even Number |
ואמנם מספר הזוג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Whatever is known concerning to it, is known in the book of the Elements. | כבר ידעת בספר היסודות ממנו מה שידעת | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Properties that are necessary to its terms will be referred to: | ונרמוז לך אל סגלות יתחיבו למדרגותיו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
מהן שאתה תמצא מקובץ מדרגותיו שוה למרבע מספר המדרגות מצורף אליו צלעו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כמו שאתה כאשר תתחיל מהשנים ותצרף אליהם הארבעה יהיו ששה והוא כמו מרבע מספר המדרגות שהם שנים וכמו צלעו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכמו שאתה כאשר תתחיל מהשנים ותצרף אליהם הארבעה והששה יהיו שנים עשר והוא כמו מרבע השלשה וכמו צלעו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומסגלותיו שכל זוג יוסיף על הראשון מהנפרדים באחד הנה זה הזוג שוה למקובץ חלקי מרבע זה הראשון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כמו הארבעה אשר הוא מוסיף על השלשה שהוא ראשון באחד ומרבע השלשה תשעה ולהם מהחלקים שני חלקים תשיעית ושלישית ומקובצם שוה אל הארבעה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וג"כ הששה יוסיף באחד על החמשה שהוא נפרד ראשון ומרבע זה הראשון עשרים וחמשה ולו מן החלקים חומש וחומש החומש לא זולתם והגעתם ששה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואמנם אם יהיה הזוג באופן שכאשר נגרע ממנו שלשה ישאר נפרד ראשון הנה זה הזוג מורכב מחלקי כפל זה הנפרד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כמו השמנה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|
שהם כאשר נגרע מהם שלשה נשארו חמשה וכפלם עשרה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|
ולהם חצי וחומש ועשירית ומקובץ זה שמנה רצוני מקובץ החמשה והשנים והאחד |
Types of Even and Odd Numbers |
ונדבר עתה בסגלות מיני הזוג ומיני הנפרד | ||||||||||||
| Starting from the properties of the even number, as their categorization to types is more reasonable than the categorization of the odd numbers. | ונתחיל בסגלות הזוג לפי שחלוקתם אל מינים יותר קרובה מחלוקת הנפרדים | ||||||||||||
Types of Even Numbers |
|||||||||||||
| Starting from the properties of the even-times-even number, since it is simpler. | ונתחיל בסגלות הזוג הזוג לפי שהוא יותר פשוט | ||||||||||||
Even-Times Even |
|||||||||||||
| The quality of its production by way of doubling and other of its properties that are in the book of the Elements, are already known. | וכבר ידעת איכות התילדותו על דרך ההכפלה וסגלות אחרות מהסגלות אשר לו בספר היסודות[4] | ||||||||||||
| Of the properties of the even-times-even number there are parts of properties that were mentioned in the Elements. | [5] מסגלות זוג הזוג מה שהם סעיפים מסגלות נזכרו ביסודות | ||||||||||||
|
שהוא אין חלק לו נקרא בשם מספר נפרד ולא זוג בלתי זוג הזוג[6] | ||||||||||||
|
ואין זוג הזוג שהוא פחות ממנו שלא ימנה אותו | ||||||||||||
|
וכל זוג זוג הנה מרבעו זוג הזוג | ||||||||||||
|
וכאשר חוסר ממנו הזוג הראשון והוא שנים יצא זוג הנפרד[7] | ||||||||||||
|
כמו השמנה שנחסר מהם שנים ויצא זוג הנפרד והוא ששה | ||||||||||||
|
וכל זוג הזוג הנה הוא חסר וחסרונו באחד[8] | ||||||||||||
|
ומסגלות זוג הזוג שמדרגותיו נמשכות על יחס מתדמה תשברתיי[9] | ||||||||||||
|
לפי שהם נמשכות על דרך ההכפל | ||||||||||||
|
והנה לא תהיינה העדפותיהם שוות | ||||||||||||
|
אבל יהיה כל העדף שוה למועדף עליו | ||||||||||||
|
ותהיינה ההעדפות עודפות בזה שביניהם זה ההעדף בעצמו | ||||||||||||
|
ויתחיב מהיות מדרגותיו על היחס האחד שתהיינה מתיחסות כשנחתוך אותם ומתיחסות כשנוסיף עליהם על השווי[10] | ||||||||||||
|
ויתחיב שיהיה הכאת אי זה אמצעי שנקח בעצמו כהכאת אחת מהשתי פאות באחרת | ||||||||||||
|
לפי שיחס הפאה הקטנה אל האמצעי כיחס האמצעי אל הפאה הגדולה | ||||||||||||
|
ויתחיב שתהיה הכאת אחד מהשני אמצעיים באחר כהכאת אחת מהשתי פאות באחרת | ||||||||||||
|
לפי שיחס הפאה הקטנה אל האמצעי הקטן כיחס האמצעי הגדול אל הפאה הגדולה | ||||||||||||
|
והנה תהיינה המדרגות ב' ד' ח' י"ו ל"ב ס"ד | ||||||||||||
|
|
ונמצא ד' בעצמו כמו ב' בח' | ||||||||||||
|
|
וח' בעצמו כמו ב' בל"ב וד' בי"ו | ||||||||||||
|
|
ונמצא ד' בח' כמו ב' בי"ו | ||||||||||||
|
|
וח' בי"ו כמו ד' בל"ב וב' בס"ד | ||||||||||||
|
ולפי שהיו מספרי זוג הזוג מסודרים על יחס מתדבק יחויב שיהיה למרבעים ולמעקבים מהם סדר באשר המרבע יהיה השלישי אליו מרבע והמעקב הרביעי אליו מעקב וילך על הדרך הזה | ||||||||||||
|
ומסגלותיו שהמספרים השלמים יתילדו ממנו[11] | ||||||||||||
|
אמנם המספרים הנאהבים הנה הם המספרים אשר יתרכבו כל אחד מחלקי חברו כמו שיתרכב חברו מחלקיו | ||||||||||||
|
כמו מאתים ועשרים ממאתים ושמנים וארבעה | ||||||||||||
|
|
כי למאתים ושמנים וארבעה חצי והוא קמ"ב | ||||||||||||
|
|
להם רביעית והוא ע"א | ||||||||||||
|
|
ולהם חלק מאחד ושבעים והוא ד' | ||||||||||||
|
|
ולהם חלק ממאה וארבעים ושנים והוא ב' | ||||||||||||
|
|
ולהם חלק ממאתים ושמנים וארבעה והוא א' | ||||||||||||
|
|
ואומר נקבץ אלה החלקים יהיו ר"כ | ||||||||||||
|
| ||||||||||||
|
|
ואמנם חלקי ר"כ הנה להם החצי והוא ק"י | ||||||||||||
|
|
ורביעית והוא נ"ה | ||||||||||||
|
|
וחמישית והוא מ"ד | ||||||||||||
|
|
ועשירית והוא כ"ב | ||||||||||||
|
|
וחלק מאחד עשר והוא עשרים | ||||||||||||
|
|
וחלק מעשרים והוא י"א | ||||||||||||
|
|
וחלק מכ"ב והוא עשרה | ||||||||||||
|
|
וחלק ממ"ד והוא ה' | ||||||||||||
|
|
וחלק מנ"ה והוא ד' | ||||||||||||
|
|
וחלק מק"י והוא ב' | ||||||||||||
|
|
וחלק מר"כ והוא א' | ||||||||||||
|
|
וכאשר תקבץ אלה החלקים יהיו רפ"ד | ||||||||||||
|
ואין לאחד מהם מן החלקים זולת מה שזכרנו | ||||||||||||
|
ואופן התילדותם | ||||||||||||
|
הוא כאשר תקבץ זוג הזוג והאחד עמהם ויקובץ מספר ראשון בתנאי שיהיה כאשר יתוסף עליו האחרון שבהם או יחוסר ממנו אשר לפניו יהיה המגיע אחר התוספת והמגיע אחר החסרון מספר ראשון | ||||||||||||
|
הנה הכאת המגיע הנוסף עליו במגיע המחוסר אחר כן הכאת העולה באחרון שבמקובצים הוא מספר אשר לו אוהב | ||||||||||||
|
ואוהבו הוא המספר אשר יהיה מתוספת הכאת מקובץ הנוסף והמחוסר הנזכרים באחרון שבמקובצים על המספר הנמצא ראשונה אשר הוא אוהב והם נאהבים[12] |
Even-Times Odd |
ואמנם סגלות זוג הנפרד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Whatever is known concerning to it, is known in the book of the Elements. | הנה כבר נודע בספר היסודות ממנו מה שנודע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| It is clear from their rule: | ונתבאר מכללם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שהוא לא ימנהו זוג אלא במספר נפרד ולא נפרד אלא במספר זוג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וחלקו הזוג נקרא בשם הנפרד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כמו השנים שהם שלישית הששה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וחלקו הנפרד נקרא בשם הזוג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כמו השלשה שהוא חצי הששה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ושתוספת הזוג הראשון והוא השנים עליו יוציא זוג הזוג[13] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ודע שהתילדותו מכפל הנפרדים הנמשכים בשנים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה תדע מזה שהנופל בין מדרגה ובין המדרגה הנמשכת אליה כפל הנופל אשר היה בנפרדים הטבעיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ויהיה העדף מדרגותיו בארבעה ארבעה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ושהוא אין נגדר בו ולא מעקב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כי כל נגדר וכל מעקב אם נפרד ימנה בנפרד במספר נפרד ואם זוג ימנה בזוג במספר זוג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכבר ידעת זה לפי שהיה ההעדף בארבעה ארבעה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וההתחלה אם מהשנים ואם מהששה כפי מה שיתבאר הענין בו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|
והשנים כאשר נוסיף עליהם ארבעה יהיו ששה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|
וכאשר נוסיף על הששה ארבעה יהיו עשרה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|
וכאשר נוסיף עליהם ארבעה יהיו ארבעה עשר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|
וכאשר נוסיף עליהם ארבעה יהיו שמנה עשר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|
וכאשר נוסיף עליהם ארבעה יהיו שנים ועשרים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה שבנו אל השנים חוזרים בסבוב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
יחויב שיהיה סבוב אחד האחרים זולת אלו ויחויב שיהיה כל דומה אל הראשון באחדים או גלגל הנקרא ציפרי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר תשים התחלת המדרגות מהששה והששה להם שליש והם הנקראים על שם שלשה הנה כאשר תתחיל אחר הששה תמצא לשלישי אחריו והוא שמנה עשר שליש אמתי ולשלישי כאשר תתחיל אחר השמנה עשר והוא השלשים שליש אמתי ועל הדרך הזה אל בלתי תכלית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואחר הששה העשרה והחלק שלהם נקרא בשם הנפרד אשר אחר השלשה והוא החמשה כי לעשרה חומש אמתי והנה כאשר תתחיל אחר העשרה תמצא הנגזר לו השם מזה המספר והוא החמישי לו חומש אמתי ועל הדרך הזה עד מה שתרצה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והמספר אשר אחר העשרה הוא הארבעה עשר וחלקו נקרא בשם הנפרד הנמשך אל החמשה כי הנה לו שביעית והנה נמצא השביעי כאשר תתחיל אחריו על הדרך הזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומסגלת אלו המדרגו' שמקובץ השנים והוא הראשון שבזוג הנפרד עם כל מדרגה תהיה נקראת על שם מספר מרובע יוציא מספר מרבע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כמו קבוצם עם הרביעי מהם והוא ארבעה עשר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|
ועם התשיעי מהם והוא ארבעה ושלשים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנמשך אל שנים והוא הששה והוא זוג הנפרד השני כאשר יקובץ אל מספר כל מדרגה מתחלת מן האחד שתהיה נקראת על שם מספר מרבע הנה יהיה המקובץ מרבע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כמו הששה עם הרביעי והוא עשרה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|
ועם התשיעי והוא שלשים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומזה שהכאת הנקרא בשם כל מדרגה בארבעה יהיה כאשר תגרע ממנו המספר הראשון יהיה מספר אותה המדרגה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
המשל בזה שהבית הרביעי נקרא בשם ארבעה וכאשר נכפל בארבעה יהיה ששה עשר תפיל מהם המספר הראשון והוא שנים הנה יהיה ארבעה עשר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכבר אפשר לך שתהפך זה ותאמר שכל מספר מהם כאשר תוסיף עליו שנים ותחלק על ארבעה הנה מה שיצא הוא מספר מדרגתו מן הראשון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומזה שכפל הכאת מספר המדרגות בעצמם שוה למקובץ מספרם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה תהיינה המדרגות ארבעה וכפל הכאתם בעצמם שנים ושלשים והנה זה מקובץ שנים ששה עשרה ארבעה עשר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומזה שמקובץ הראשון והשני מעקב ח' ואתה תדענו ותדע מדרגתו ממה שכבר ידעת ועוד מעקב מעקבו ועל הדרך הזה[14] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה נבנה מזוג הנפרד הנמשכים מרבע ששה בששה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומסגלות זה הלוח המרבע שאחדי ראשית התחלת כל שורה ברוחב כמו אחדי הסוף | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם יהיה באחד מהם גלגל הנקרא ספרא הנה באחר גלגל גם כן | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומהם שמקובץ שני קצוות הקטר האחר שוה למקובץ שני קצות הקטר האחר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כמו ב' עם קמ"ב שהם שני קצות הקטר וכ"ב עם קכ"ב והם קצות הקטר האחר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומהם שמקובץ קצות הקטר נגדר לעולם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומהם שכל שני מספרים מרחקם משני קצות הקטר מרחק אחד הנה מקובצם שוה למקובץ שתי קצות הקטר והוא לזה נגדר גם כן | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומזה שתוספת כל שורה על התחלת זאת השורה אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כי תוספת כ"ב על מ"ו כתוספת מ"ו על ע' |
Even-Times-Even-Times Odd |
ואמנם עניני זוג הזוג והנפרד הנה נדבר בהם |
|
ונאמר שהוא דומה לזוג הנפרד באשר הוא איננו מקבל ההחצות ההולך בהדרגה בהתמדה עד האחד מזולת שבר |
|
וידמה לזוג הזוג באשר הוא לא יחצה החצותו הראשון אל שני נפרדים ולא יעמד החצותו אצל חלוקה אחרת |
|
ואמנם התילדותו הנה הוא מהכאת זוג הזוג המתחילים מן הארבע בנפרדים הנמשכים |
|
וכל מה שהיה זה הזוג יותר רב יהיה קבלתו להחצות יותר |
|
וכבר יהיו בו הנוסף והחסר והשלם |
|
כי השמנה וששים מספר חסר והוא מכללו |
|
ואמנם השלם הנה כמו השמנה ועשרים |
|
והנוסף בו הרבה כמו השנים עשר |
|
וכבר יפלו בו המרבעים ג"כ |
|
והתילדות אלו המרבעים אשר יפלו במספריהם |
|
הוא שנכה הזוג הראשון בנפרד הראשון עד שיהיה ששה והנה הוא גדר המרבע הראשון |
|
אח"כ נכה אותו בנפרד השני עד שיהיה עשרה והנה הוא גדר המרבע השני |
|
ועל הדרך הזה |
|
וכאשר תגרע הבית מאשר ימשך אליו יצא לך זוג הזוג |
|
כמו השנים עשר מהעשרים |
|
וזה במה שהתילדותו מהכאת הארבעה בנפרדים |
|
וכמו הארבעה ועשרים מן הארבעים |
|
וזה במה שהתילדותו מהכאת השמנה בנפרדים |
| This is what is said on the properties of the types of the even-times-even numbers that are affected by the properties of the odd number. | והנה זה מה שנאמר אותו בסגלות מיני זוג הזוג ונעתק אל מיני סגלות הנפרד |
The Two - is it an even-times-even number or an even-times-odd number? |
|
| The discussion on the first of the numbers, which is two, still remains: is it an even-times-even number, or an even-times-odd number? | וכבר נשאר עלינו הדבור בראשון שבמספרים והוא השנים האם הוא זוג הזוג או זוג הנפרד |
|
וכבר יחשב מצד שהוא לא יכלה בו ההחצות אל זוג שהוא זוג הנפרד |
|
והעבירו קצתם שיהיה זוג הזוג וזוג הנפרד יחד ושיהיה התחלה לשניהם |
[According to the author]:
|
והנראה אלי שזוג באמת הוא המספר המתחלק אל הנפרד אצל ההחצות |
|
וזוג הנפרד באמת הוא המתחלק אל הנפרד אצל ההחצות |
|
והנה הזוג הזוג הוא אשר חציו זוג וכל חצי יחצה אותו זוג זולת האחד |
|
ולא ימלט מהחצות זוג |
|
וזוג הנפרד הוא אשר חציו נפרד ולא יחצה |
|
והנפרד יהיה מספר או יהיה אחד מצד שלא יחלק בשני חלקים |
| ? | והזוג לא יהיה ולא מספר |
| אחר זה הנה ראוי שלא נקפיד בקריאת השמות | |
|
ואם ירצה אחד שנשים השנים ראויים לשני השמות יחד |
|
הנה ראוי שנשים גדר זוג הזוג שהוא אשר לא יחצה אל מספר נפרד |
|
וכן הם השנים אמנם החלוקה לא תהיה על דרך היושר |
|
ואם רצה שנוציא השנים משני השמות יחד |
|
הנה ראוי שנשים גדר זוג הזוג שהוא הנחצה אל מספר זוג |
|
וגדר זוג הנפרד שהוא אשר יחצה אל מספר נפרד |
|
ולא יהיו השנים ראויים אל אחד משני השמות עם יושר החלוקה |
Types of Odd Numbers |
|
| Now the properties of the types of odd numbers will be discussed: | ונדבר עתה בעניני מיני הנפרד |
| Some of the odd numbers are prime and some are composite. | והנפרד ממנו ראשון וממנו מורכב |
| The composite may be relatively prime. | והמורכב כבר יהיה ראשון בהקש אל זולתו |
| All this is already known. | וכבר ידעת כל זה |
Composite Numbers |
|
| When wishing to extract the terms of the composite numbers, one should return to the tables of the successive odd numbers: | וכאשר תרצה שתוציא מדרגות המורכבים בעצמם הנה תשוב אל לוחות המספרים הנפרדים הנמשכים |
|
והנה תמצא כל שלשה אחר השלשה מורכב |
|
וכל חמשה אחר החמשה מורכב |
|
וכן אל בלתי תכלית |
|
דמיון הראשון הט' והט"ו והכ"א |
|
דמיון השני הט"ו והכ"ה והל' |
|
וכמו כן כאשר תתחיל מן השבעה והתשעה על הדרך הזה |
|
ותמצא שם דבר והוא שהשלשה מהם ימנה |
|
המורכב הראשון אשר במדרגותיהם במספר ראשון הנפרדים והוא בעצמו כמו התשעה |
|
והשני בנפרד אשר ימשך אליו כמו החמשה |
|
והשלישי בנפרד השלישי כמו השבעה[15] |
|
והחמשה ג"כ ימנה |
|
אשר ימשך אליו בראשון בנפרדים והוא השלשה כמו החמשה עשר |
|
והשני בעצמו כמו החמשה ועשרים |
|
והשלישי במה שאחריו כמו החמשה ושלשים כי הוא ימנה אותם בשבעה[16] |
Relatively Prime Numbers |
|
|
ואמנם המורכב בעצמו וראשון אצל זולתו |
|
הנה הוא כל מרבע ראשון בהקש אל מרבע ראשון אחר מאלו הנפרדים הנמשכים[17] |
| This is what is said about the properties of the even and the odd numbers. | והנה זה מה שנאמר אותו בעניני הזוג והנפרד |
Superabundant / Deficient / Perfect Numbers |
|
| Another categorization of the number: some number are superabundant, some are deficient, and some are perfect. | והמספר חלוקה אחרת ממנו נוסף וממנו חסר וממנו שלם |
| All this is already known, as well as the quality of the production of the perfect number from the even-times-even numbers. | וכבר ידעת כל זה וידעת איכות התילד המספר השלם מזוגי הזוגות[18] |
| The perfect number is an even number only, because it is produced from the multiplication of an odd number by an even number. | ודע שהמספר השלם לא יהיה אלא זוג לפי שהוא אמנם נתילד מהכאת נפרד בזוג |
|
ונזדמן שהנופל ממנו באחדים אחד והוא ששה |
|
ובעשרות אחד והוא שמנה ועשרים |
|
ובמאות אחד והוא תצ"ו |
|
ובאלפים אחד והוא שמנה אלפים וקכ"ח |
|
וכמו כן בכל מין אחד |
|
לא ימלט מאחדים הם ששה ושמנה ואם לא יתחיב אצל הנסיון בהם שיהיו חוזרים חלילה |
|
ומסגלות המספר השלם שכאשר הוכה בשמנה ונוסף עליו אחד יהיה נגדר |
|
וכאשר יחלק גדרו על ארבעה ויתוסף על מה שיתקבץ רביע יהיה זוג הזוג אשר הוכה בכפלו זולת אחד עד שיצא זה המספר השלם |
|
המשל בזה ששה בשמנה נוסף עליו אחד הוא מ"ט |
|
וגדרו שבעה ורביעיתו אחד ושלשה רביעיו' וכאשר יתוסף עליו רביע יהיה שנים והוא זוג הזוג |
|
|
אשר נפלה ההכאה בכפלו זולת אחד כאשר יצא ששה[19] |
| The superabundant and the deficient numbers are as explained in every chapter [?] | אמנם המספר הנוסף והחסר הנה יהיו כמו שכבר נתבאר בכל שער |
|
והנה בהוצאת השלם והחסר והנוסף בחינה נפלה לקצת האנשים |
|
והיא שכל זוג הזוג כאשר הוכה במספר ראשון איך שיהיה |
|
אחר שיהיה זוג הזוג יותר מחצי זה הראשון בחצי אחד הנה המתקבץ ממנו לעולם מספר שלם |
|
כמו השנים בשלשה |
|
|
והארבעה בשבעה |
|
ואם יהיה יותר מחציו ביותר מחצי אחד הנה המתקבץ ממנו לעולם מספר נוסף |
|
ואם יהיה פחות מחציו איך שיהיה הפחת הנה המספר חסר |
|
משל הראשון הנה ארבעה בחמשה |
|
ומשל השני הארבעה באחד עשר |
|
וכל מספר מהמספרים השלמים הוכה במספר ראשון ולא ימנה זה המספר הראשון זה המספר השלם |
|
כמו הששה כאשר הוכה בשבעה ונתחדש מ"ב |
|
|
ולו מן החלקים החצי והוא כ"א |
|
|
ושליש והוא י"ד |
|
|
והששית והוא ז' |
|
|
והשביעית והוא ו' |
|
|
וחלק מי"ד והוא ג' |
|
|
וחלק מכ"א והוא ב' |
|
|
וחלק ממ"ב והוא א' |
|
וכלל זה נ"ד |
|
הנה מוסיף על מ"ב בי"ב והוא כפל ששה |
|
וכל מספר ימנהו שנים הנה הוא חסר לעולם |
|
וכל המספרים הראשוני' חסרי' בלי ספק |
|
וכל זוגי הזוג חסרים באחד |
|
וכל מספר זולת הששה ימנוהו השנים והשלשה הנה הוא נוסף לעולם |
|
וכל מספר ימנה אותו השנים ושני מספרים יהיה קריאת מקובצם עומדת מקום השליש רצוני שיהיה אחריתם כמו השליש רצוני שיהיה החבור משני יחסי חלקיהם בנכח המוסיף שליש כמו מקובץ שני יחסי המוסיף חומש והמוסיף שביעית אשר הוא לנכח המוסיף שלישית הנה הוא נוסף לעולם |
|
כמו השבעים כי הוא לפי שהוא נמנה עם השנים והחמשה והשבעה יהיה נוסף לעולם |
|
וכל זוג נפרד נמנה עם השנים והחמשה והשבעה יהיה נוסף |
|
וכל זוג נפרד מורכב מנפרד מורכב כמו השמנה עשר והשלשים הנה הוא נוסף תמיד |
|
ואם יהיה מורכב מנפרד ראשון הנה הוא חסר |
|
וכבר ימצאו בזוג הזוג והנפרד חסר ונוסף ושלם |
|
דמיון הנוסף ששה ושלשים |
|
ודמיון החסר ארבעים וארבעה |
|
ודמיון השלם שמנה ועשרים |
|
המספר הנפרד לא יהיה שלם כמו שידעת אבל יהיה חסר |
|
ולא יהיה נוסף אלא שיהיה מורכב מארבעה נפרדים נמשכים על הסדר הטבעי |
|
כמו מה שראשיתו שלשה אח"כ חמשה אח"כ שבעה אח"כ תשעה ומורכבם הוא תשע מאות וארבעים וחמשה והוא המספר הראשון שבנפרדים נוסף בשלישית ואם יניח זה ההמשך לא יתחיב שיהיה נוסף |
| The end of the discussion on this manner of arithmetic - moving on to the manner by which the relation of a number to a number is examined. | ונחתום הנה הדבור בזה האופן מחכמת המספר ונעתק אל האופן אשר יבחן ממנו בצרוף מספר אל מספר |
Book Two |
המאמר הב' |
|---|---|
Ratios |
|
| The number is examined by examination from the aspect of its attention to itself and to the properties that are necessary for it, to what is a number and what is a type of number. | כבר יעויין במספר עיון מצד ההשגחה בעצמו ובעניינים המתחייבים לו במה שהוא מספר ומה שהוא מין מספר |
| It is examined from other aspects, among them from the aspect of being related to another number. | וכבר יעויין בו מצדדים אחרים מהם מצד היותו מצטרף אל מספר אחר |
| This other number is either other from it by number and not by species, or part of the species, so the relation is a relation of changing. | וזה המספר האחר אם יהיה אחר ממנו במספר לא במין או חלק המין יהיה הצרוף צרוף השנוי |
| Every two changing terms - one of them is greater and the other is less | וכל שני משתנים הנה אחד מהם מוסיף והאחר חסר |
| When one knows the properties of the greater to the less, he knows the properties of the less to the greater, according to what the evenness requires in relation. | וכאשר תדע עניני המוסיף אצל החסר תדע עניני החסר אצל המוסיף כפי מה שיחיבהו היושר בהצטרפות |
| The greater is either simple or not simple. | והמוסיף אם פשוט ואם בלתי פשוט |
|
והפשוט אם כפל ואם כפלים ואם מוסיף בחלק או חלקים |
|
והמורכב הוא המוסיף בכפל והחלק או המוסיף בכפל והחלקים או המוסיף בכפלים והחלק או המוסיף בכפלים והחלקים |
|
והחלקים כונתנו בהם מה שהוא יותר מכפל אחד או חלק אחד ואם היה שני כפלים או שני חלקים |
| As for the less, the custom has spread to indicate it by sub-, as saying subsuperparticular | והחסר הנה כבר פשט המנהג שיורו עליו באשר הוא אשר תחת ככה כאמרם אשר תחת המוסיף חלק |
| Sometimes its name is derived from the name of the number of the multiples, such as the third, the quarter, and the twelfth; and sometimes it is called by two ratios, as saying half the sixth, or fifth of a tenth. | ולפעמים יהיה נגזר לו שם משם מספר הכפלים כמו השליש והרביע והחלק משנים עשר ולפעמים יקרא בשני יחסים כאמרם חצי הששית חומש עשירית |
Simple Ratios |
|
Multiple Ratio |
|
|
והנה הנכפל הראשון הוא הנכפל השניי והוא אשר התוספת בו בדמיון האחר |
|
והתחלתו במספרים מן האחד והשנים |
|
ויתוסף החסר על סדר המספרים הנמשכים |
|
והמוסיף והוא הכפל על סדר הזוגות הנמשכים בהעדף שנים שנים |
|
אח"כ הנכפל השלישיי והוא אשר התוספת בו בשני דמיונים |
|
והתחלתו מהשלשה והאחד |
|
ויתוסף החסר על סדר המספרים הנמשכים |
|
והנוסף בשלשה שלשה |
|
כמו שלשה וששה ותשעה ושנים עשר |
| According to this way the less are added one by one in all the multiple ratios and the greater by the number of the multiples. | ועל הדרך הזה יתוסף החסר בכל היחסים בעלי הכפל באחד אחד והנוסף במספר הכפלים |
| The beginning of the less is from 1. | ותהיה התחלת החסר מן האחד |
| The beginning of the greater from the number by which the number of the multiple is denominated [= the multiplier]. | והתחלת הנוסף מהמספר אשר על שמו יקרא מספר הכפלים |
Superparticular Ratio |
|
|
והראשון אשר במוסיף חלק הוא המוסיף על האחר בכמו חציו |
|
והתחלתו מהשלשה והשנים |
|
ויתוסף החסר על סדר הזוגות הנמשכים לפי שיש להם חצי |
|
והמוסיף בשלשה שלשה |
|
כמו השנים עם השלשה אח"כ הארבעה עם הששה אח"כ הששה עם התשעה |
|
ואחר המוסיף חצי הוא המוסיף שליש |
|
והתחלתו מן הארבעה והשלשה |
|
ויתוסף החסר בשלשה שלשה |
|
כמו השלשה והששה והתשעה |
|
והמוסיף בארבעה ארבעה |
| According to this way the matter proceeds always by this order. | ועל הדרך הזה ילך הענין תמיד על זה הסדר |
Table of Multiple and Superparticular Ratios |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Setting a square table with lines, starting from one, the beginnings of its lines are added lengthwise and breadthwise by the sequence of the natural numbers | והנה כאשר נרשום לוח בעל טורים מרובע יתחיל מן האחד ויתוספו ראשי שורותיו בארך וברחב על סדר המספרים הטבעיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| By this way these ratios are established in it, as well as other propositions that are derived from them. | ועל הדרך הזה תניח בו אלה היחסים ומשפטים אחרים יוצאים מהם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה יהיה זה הלוח הבעל טורים עשרה על עשרה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה נמצא השורה השנית על יחס הכפל לשורה הראשונה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והשלישית על יחס השלשה כפלים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| By this way the excess is found as is said. | ועל הדרך הזה נמצא ההעדף כפי מה שנאמר לך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונמצא השורה השלישית אל השנית על יחס המוסיף חצי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והרביעית אל השלישית על יחס המוסיף שליש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והחמישית אל הרביעית על יחס המוסי' רביע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| According to this way the matter proceeds always. | ועל הדרך הזה הולך הענין תמיד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The excess is found as is said: | ונמצא ההעדף כפי מה שנאמר לך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונמצא תוספת השורה השנית על השורה הראשונה יתחלף במספר ואם לא יתחלף ביחס | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונמצא תוספת הבית הראשון ממנו על הבית הראשון מהשורה הראשונה באחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותוספת הבית השני ממנו על הבית השני מהראשון בשנים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| According to this way by the order of the successive numbers. | ועל הדרך הזה על סדר המספרים הנמשכים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועל הדרך הזה ענין כל בית אצל הקודם אליו[20] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונמצא זה כאשר יוקש בין השלישי והראשון בכל סדור על סדר הזוגות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כי הנה נמצא הראשון מכל שלישי יוסיף על ראשון מכל ראשון בשנים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והשני בארבעה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והשלישי בששה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועל הדרך הזה[21] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואמנם תוספת הבית הראשו' מ[הר]ביעי על הבית הראשון מכל ראשון הנה הוא בשלשה שלשה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותוספת השני מן הרביעי על השני מן הראשון בששה ששה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועל הדרך הזה תוספת כל בית יוסיף על תוספת הבית בשני צדדין בשלשה שלשה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תוספת רביעי על השני וביניהם שורה אחת כתוספת השני על הראשון ביחס | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותוספת הששי על השלישי וביניהם שתי שורות כתוספת השלישי על השני ביחס ועל הדרך הזה[22] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה תמצא כל מספר ממספרי הקטר מרבע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כמו הד' והט' והי"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונמצא מקבץ כל שני מרבעים נמשכים ומקבץ השני שטחים אשר יפלו ביניהם על האלכסוניות מרבע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כמו מקבץ הד' והט' עם הו' וו' שעולה כ"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונמצא מקבץ כל שני מרבעים נמשכים על מקבץ השני שטחים מוסיף באחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה יחויב שיהיה כפל מקובץ כל שני מרבעים נמשכים מחוסר ממנו אחד מרבע[23][24] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונמצא הכאת כל מספר משורה במספר משורה אחרת שוה להכאת בן גילו בבן גילו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כמו הב' והוא השני מן הראשונה בכ' והוא האחרון מן השנית אשר הוא כמו הד' אשר הוא השני מן השנית בי' אשר הוא האחרון מן הראשונה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונמצא הכאת כל מספר ממספרי הקטר בבן גילו מהצד האחר מזה הקטר כמו בני גילם אחד מהם באחר רצוני מן הקטר האחר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כמו הכאת הא' בק' אשר הוא כמו הכאת הי' בי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וג"כ הכאת ד' בפ"א אשר הוא כמו הכאת י"ח בי"ח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועל הדרך הזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| As for the other ratios, before examining them in this table, the quality of rule of investigating their first numbers will be referred to. | ואמנם היחסים האחרים הנה אנחנו קודם שנבחין אותם מזה הלוח הבעל טורים נרמוז אל איכות ההנהגה בדרישת מספריהם הראשונים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Their properties will be determined, then their examination in this table will be alluded to. | ונרמוז אל ענינים מסגלים להם אח"כ נרמוז אל בחינתם מזה הלוח |
Superpartient Ratio |
|
| The superbipartient ratio or the superpartient ratio is sometimes absolute and sometimes not absolute. | ונאמר אם יחס המוסיף בשני חלקים או המוסיף בחלקים הנה לפעמים יהיה גמור ולפעמים לא יהיה גמור |
| The absolute, meaning that which is not reduced to the superparticular ratio. | והגמור רצוני מה שאיננו חוזר אל יחס דמיון וחלק |
|
כמו חזרת המוסיף בשני ששיות אל המוסיף שליש |
|
והמוסיף בשני רביעיות אל המוסיף חצי |
|
כל מוסיף בשני חלקים נקראים בשם זוג |
|
וחזרת המוסיף בשלשה ששיות אל החצי אחר כן |
Such as the superbiquintan 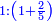 and the supertriquartan and the supertriquartan 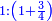 are the [absolute ratios]. are the [absolute ratios].
|
ואבל כמו המוסיף בשני חמישיות והמוסיף בשלש רביעיות הוא הבלתי גמור |
| There is no common order for the absolute ratios, thus any one who measures should examine the sequence. | ולא ימצא לגמור סדור משותף בו אבל יצטרך כל שוער להבחין הסדור |
When it is taken in actu, the order of the realization of its first number is such that the first is that by which the part of the numbers is denominated, so that two is added to it if there are two parts or three if there are three parts.
|
ואמנם כאשר ילקח בשלוח[25] הנה הסדר בהגעת מספרו הראשון הוא שיגיע הראשון אשר בשמו יקרא זה החלק מן המספרי' כדי שנוסיף עליו אם הוא שני חלקים הנה שנים ואם היה שלשה חלקים הנה השלשה |
|
המשל בזה אם היה התוספת בשני שלישיות נניח שלשה ונוסיף עליהם שנים ויהיו חמשה ותהיה התחלתם משלשה וחמשה |
|
ואם יהיה התוספת בשלשה רביעיות נניח ארבעה ונוסיף עליהם שלשה ויהיו ארבעה ושבעה והיא מתחלתם |
|
והנה תמצא המספרים החסרים ביחס הדמיון ושני חלקים |
|
אם ביחס הדמיון ושני שלישיות |
|
הנה החסרים מוסיפים בשלשה |
|
והנוספים בחמשה |
|
|
עד שיהיו שלשה וחמשה אח"כ ששה ועשרה אח"כ תשעה וחמשה עשר |
|
ואמנם ביחס הדמיון ושתי רביעיות והוא בלתי גמור |
|
הנה תמצא החסרים יוסיפו בארבעה ארבעה |
|
והנוספים בששה ששה |
|
|
באלו מספרם על הקש ארבעה וששה ושמנה ושנים עשר |
|
ועל הדרך הזה החסר כמו עצמו והנוסף כמו עצמו |
|
וכפי זה הוא הסדר במוסיף שתי חמישיות |
Comparing [the superbipartient ratios] to each other [![\scriptstyle\left[1:\left(1+\frac{2}{n}\right)\right]:\left[1:\left(1+\frac{2}{n+1}\right)\right]](/mediawiki/images/math/b/1/5/b1597dbddfa7136a5e5ddd7e45afc6c8.png) ]: ]:
|
ואמנם הקש קצתם אל קצת |
|
רצוני הקש המוסיף שתי שלישיות אל המוסיף שתי רביעיות אח"כ המוסיף שתי חמישיות |
|
הנה החסרים יוסיפו באחד אחד |
|
והנוספים ג"כ יוסיפו באחד אחד[26] |
|
וכאשר תבחין הגמורים בזה היחס יהיו על סדר הנפרדים הנמשכים |
|
כמו החמשה אל השלשה והוא המוסיף בשתי שלישיות |
|
והשבעה אל החמשה והוא המוסיף בשתי חמישיות |
|
והתשעה אל השבעה והוא המוסיף בשתי שביעיות |
Comparing between the supermultiplepartient [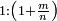 ]: ]:
|
ואמנם ההקש בין רבי החלקים |
|
כמו המוסיף בדמיונו ושלשה רביעיות |
|
הנה יוסיפו החסרים מהם והמוסיפים מהם על ההקש הנזכר |
|
|
עד שיהיו ארבעה ושבעה אח"כ שמנה וארבעה עשר |
|
ועל הדרך הזה תוספת שלש חמישיות |
|
|
יהיה חמשה ושמנה ועשרה וששה עשר |
|
ויהיה התיחסות מה שביניהם כפי מה שנאמר בראשונים |
|
כמו ארבעה ושבעה אח"כ חמשה ושמנה אח"כ ששה ותשעה |
| The absolute ratios have unordered properties that should be drawn by examination for each category. | וימצאו לגמורי' סגלות בלתי מסודרות אלא בשער שער יש להוציא אותם עם הבחינה |
Compounded Ratios |
|
Multiple Superparticular Ratio |
|
| When wishing to find the first number in the [multiple] superparticular ratio: | וכאשר נרצה שנמצא ראשון המספרים ליחס הדמיון והחלק[27] |
| Taking that by which the parts are denominated, as two for a half, three for a third, then multiplying this number by two and adding one to it.
|
הנה נקח אשר על שמו יקראו החלקים כמו השנים לחצי והשלשה לשליש ונכפול זה המספר בשנים ונוסיף עליו אחד |
|
כמו הכפל וחצי כי התילדותו מכפל השנים בשנים והתוספת אחד עליו ויהיו שנים וחמשה |
|
והכפל ושליש שהתילדותו מכפילת השלשה בשנים והתוספת אחד עליו ויהיו שלשה ושבעה |
|
והכפל ורביע שיהיו ארבעה ותשעה |
|
ונמצאו המספרים בראשון[28] |
|
יתוסף החסר בשנים שנים על סדר הזוגות הנמשכים |
|
ויתוסף המוסיף בחמשה חמשה |
|
עד שיהיה במוסיף כפל וחצי שנים וחמשה אח"כ ארבעה ועשרה אח"כ ששה וחמשה עשר |
|
ונמצא המספרים בשני והוא יחס הכפל והשליש |
|
יתוספו החסרים בהם בשלשה שלשה |
|
והמוסיפים בשבעה שבעה |
|
כמו שלשה ושבעה אח"כ ששה וארבעה עשר ותשעה ואחד ועשרים |
|
ונמצאו המספרים בשלישי והוא יחס הכפל ורביע |
|
יתוסף החסר בהם בארבעה ארבעה |
|
והמוסיף בתשעה תשעה |
|
עד שיהיו על משך ארבעה ותשעה אח"כ שמנה ושמנה עשר אח"כ שנים עשר ושבעה ועשרים |
| As a rule: the addition of the less and the greater is according to their first number. | ובכלל הנה תוספת החסר והנוסף יהיה על מספרם הראשון |
The relation between their terms, meaning between the double sesquialter ratio and the double sesquitertian ratio [and the double sesquiquartan ratio] [![\scriptstyle\left[1:\left(2+\frac{1}{n}\right)\right]:\left[1:\left(2+\frac{1}{n+1}\right)\right]](/mediawiki/images/math/6/d/7/6d72c1d885f6fce8719102ff81d49e12.png) ] ]
|
ואמנם ההתיחסות במה שבין מדרגותיהם רצוני התיחסות מה שבין הכפל והחצי ובין הכפל והשליש |
|
הנה החסרים יתוספו באחד אחד |
|
והמוסיפים בשנים שנים כפי ההכפלה |
|
עד שיהיו שנים וחמשה שלשה ושבעה ארבעה ותשעה ועל הדרך הזה |
| Thus, the rule of the addition follows the successive odd numbers. | וילך מנהג התוספת על הנפרדים הנמשכים |
Multiple Superpartient Ratio |
|
|
ואמנם יחסי הכפל והשני שלישיות הנה ראוי שנעשה בתולדתם מה שכבר עשינו אותו אלא שאנחנו נוסיף תמורת האחד שנים |
|
ונתחיל אם ביחס הכפל והשתי שלישיות מן השלשה והשמנה |
|
וביחס הכפל והשתי רביעיות והוא בלתי גמור בארבעה והעשרה |
|
וביחס הכפל והשתי חמישיות מן החמשה והשנים עשר |
|
והנה נמצא המוסיפים ג"כ יוסיפו בשנים שנים והחסרים באחד אחד |
|
ונמצא הסדר והמנהג בשער אחד כמו סדר המספרים המונחים לשני דמיונים ושתי שלישיות ונמצא החסרים והמוסיפים יוסיפו על מספרם אלא שאנחנו נמצא מספר החסרים כמו שהיה בדמיון ושליש וכפל ושתי רביעיות וכפל ושתי חמישיות ושאר אלו[29] |
|
וכאשר נרצה הכפל והשלשה חלקים |
|
והראשון שבהם שלשה רביעיות |
|
הנה ההולדה על זה הדרך בעצמו אלא שאנחנו נוסיף למוסיף שלשה חלקים שלשה ולמוסיף ארבעה חלקים ארבעה |
|
והנה הראשון שבכפל ושלשה חלקים הכפל ושלשה רביעיות והתחלתו מן הארבעה והאחד עשר |
|
אח"כ הכפל ושלשה חמישיות והתחלתו מן החמשה ושלשה עשר |
|
ועל הדרך הזה |
|
והנה נמצא תוספת מדרגות המספרים כמו שהיה |
|
וכאשר נתנהג במה שבשער אחד נמצא החסרים והמוסיפים ג"כ יתוספו כמו עצמם |
|
ואמנם מספר החסרים יהיה כמו שהיה ומספר המוסיפים מספר אחד |
|
וכאשר נרצה יחס שלשה כפלים וחלק או שני חלקים אחרים |
|
נעשה בהולדת אלו מה שכבר עשינו אלא שאנחנו לא נכפול פעם אחת לבד אבל במספר אותם הכפלים אח"כ נעשה בחלק והחלקים מה שכבר עשינו |
|
|
|
ונמצא ראשית השלשה כפלים ושליש משלשה ועשרה |
|
וראשית שלשה כפלים ורובע מארבעה ושלשה עשר |
|
ונמצא החסרים יוסיפו באחד אחד והמוסיפים בשלשה שלשה |
|
וכאשר נקח ברחב |
|
נמצא ראשית שלשה כפלים וחצי משנים ושבעה ושנית מארבעה וארבעה עשר |
|
ונמצא ג"כ כלם יתוספו במספרם |
|
והחסר ירוץ על תוספת הזוגות הנמשכים |
|
ונמצא ראשית שלשה כפלים ושליש מהשלשה והעשרה ושנית מהששה ועשרים |
|
ונמצא השרש שמור |
|
וכאשר יבחנו השלשה כפלים והשני חלקים |
|
יהיה ראשיתם שלשה כפלים ושני שלישיות והראשון משלשה ואחד עשר |
|
וראשית שלשה כפלים ושני רביעיות מארבעה וארבעה עשר |
|
וראשית שלשה כפלים ושתי חמישיות מחמשה ושבעה עשר |
|
ונמצא ההעדף בחסרים על משך המספרים הטבעיים והמוסיפים בשלשה שלשה |
|
וכאשר נקח ברוחב |
|
נמצא ראשית שלשה כפלים ושתי שלישיות משלשה ואחד עשר ושנית מששה ושנים ועשרים |
|
והנה שמרו הסדר |
|
וכאשר נבחין השלשה כפלים ושלשה חלקים |
|
היה ראשית זה שלשה כפלים ושלשה רביעיות וראשיתם מארבעה וחמשה עשר |
|
אח"כ שלשה כפלים ושלשה חמישיות וראשיתם מחמשה ושמנה עשר |
|
ונמצא הענין על הדרך הזה |
|
וכאשר נבחין ברוחב |
|
נמצא ראשית שלשה ושלשה רביעיות מארבעה וחמשה עשר ושנית משמנה ושלשים |
|
ונמצא זה הסדור שמור |
Tables of Ratios |
|||||||||||||||||||||||||||||||||||||||||||||||||||
| We should elaborate on this as well as establish the relations of the tens [?] by tens and extract them, but we will be satisfied with this and mention indications from the aspect of the tables with which these are associated. | ולנו שנוסיף בזה ונישב ג"כ התיחסות הכלל בכלל ונוציא אותו אבל אנחנו נסתפק על זה ונזכור רמזים על צד הלוחות ישתתפו בהם אלו | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה מזה שאנחנו כאשר נעשה לוח משתי שורות | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
אחת מהן ימשכו בה הנפרדים הנמשכים מתחילים מן החמשה ויעמדו אצל אחד ועשרים | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
והשנית ימשכו בה מספרים מתחילים משלשה ויעמדו אצל אחד עשר | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
ויתבאר לנו שבמה שבין אלו יחסים | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר נבחין מה שבכל בית מהלוח הראשון מצורף לבן גילו מהלוח האחר | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה יהיה על יחס הדמיון ושתי שלישיות | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
אח"כ הדמיון ושלשה רביעיות | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
אח"כ הדמיון וארבעה חמישיות | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועל הדרך הזה | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר נבחין סדור מה שבלוח הראשון יהיו על יחס הדמיון ושני חלקים הגמור[30] | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר נבחין סדור מה שבלוח השני יהיו על יחס המוסיף חלק[31] | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר נניח תמורת הבית השני המתחיל מן הג' בית אחר מתחיל מן הב' וילך על משך המספרים אשר בטבע יהיה יחס הבית הראשון מהשורה הראשונה לבן גילו מן השנית על יחס שני דמיונים והחלק | ||||||||||||||||||||||||||||||||||||||||||||||||||
| We should extract from this the tables of the other remaining ratios, since the first column is shared by all the ratios. | ולנו שנוציא מזה הלוחות לשאר היחסים הנשארים עם היות שהלוח הראשון[32] ישתתף לכל היחסי' | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||
|
ויצא אלינו יחס הדמיון והחלק ממה שכבר ידעת מהטור השלישי והשני | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
ויחס הדמיון ושני חלקים | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
מהטור החמישי והשלישי והוא הדמיון ושתי שלישיות | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומן הטור הששי והרביעי והוא אל הדמיון ושני רביעיות | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומהטור השביעי והחמישי והוא אל הדמיון ושתי חמישיות | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועל הדרך הזה | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
|||||||||||||||||||||||||||||||||||||||||||||||||||
|
ויצא אלינו מן הלוח הטור השביעי והרביעי בהנחת שני טורים יחס הדמיון ושלשה רביעיות | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומן הטור השמיני והחמישי בהנחת שני טורים יחס הדמיון ושלשה חמישיות | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועל הדרך הזה | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
|||||||||||||||||||||||||||||||||||||||||||||||||||
|
ויצא אלינו מן הלוח התשיעי והחמישי בהנחת שלשה טורים יחס הדמיון וארבעה חמישיות | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומן הטור העשירי והששי יחס הדמיון וארבעה ששיות | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועל הדרך הזה יצא לנו יחס השני דמיונים וחלק מזה הלוח ג"כ | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם הראשון שבהם והנה הוא יחס השני דמיונים והחצי בהנחת שני טורים מהטור החמישי והטור השני | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם השני והוא יחס שני דמיונים ושליש הנה מהטור השביעי והשלישי כאשר תניח שלשה | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם השלישי והוא יחס השני דמיונים ורביע מהטור התשיעי והרביעי כאשר תניח ארבעה | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
ויצא לנו יחס השני דמיוני' והשני חלקים | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם השני שלישיות הנה מהשמיני והשלישי | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
והשני רביעיו' מהעשירי והרביעי | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
ויצא לנו יחס הדמיון ושלשה חלקים ושאר היחסים כאשר נתנהג בדרך אשר כוננו אליה | ||||||||||||||||||||||||||||||||||||||||||||||||||
Producing the Ratios from Equality |
|||||||||||||||||||||||||||||||||||||||||||||||||||
| The ancients referred to the method of production the ratios from equality and the extraction of the different ratios from the referred ratios. | וכבר רמזו הקדמוני' אל דרך התילד משווי היחסים והביא אל היחסים המתחלפים מהיחסי' הרמוז אליהם | ||||||||||||||||||||||||||||||||||||||||||||||||||
| Which is, that whichever equal numbers, out of which three will be arranged, all ratios can be produced from them by the method employed with them: | והיא כי איזה מספרים שוים יסודרו מהם שלשה אפשר שיתילדו היחסים כלם מהם בדרך נעשה בהם | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה יהיה לוח בו שלשה מספרים אח"כ שלשה מספרים אחרים ויהיו שלשה שלשה | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
ויתרבו לבחינה וההרחבה בנסיון | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
וימשך אליו ברוחב לוח אחר נחלק בחלקיו | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונאמר שאנחנו כאשר נקח הראשון ונניחהו בבית מכל טור ברחב על שהוא ראשון | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
אח"כ נחבר הראשון והשני ונסדר אותו בבית שני מהלוח השני יהיה מטור האחדים שנים | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
אח"כ נחבר הראשון והשלישי וכפל השני ונסדר אותו בבית השלישי ממנו ויהיה מטור האחדים ארבעה | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
אח"כ נשים הבית השני שרש | ||||||||||||||||||||||||||||||||||||||||||||||||||
| ? | ונחבר ממנו זה החבור ונעתיק אותו אל הבית השלישי זה ההעתק | ||||||||||||||||||||||||||||||||||||||||||||||||||
| The additions of these rubrics proceed continually, so they are four lengthwise. | וילך בהתמדה התוספת הזה באלה הבתים והנה יהיו ארבעה בארך | ||||||||||||||||||||||||||||||||||||||||||||||||||
| If happened first that the ratio of every three numbers on one line is a continuous ratio. | קרה מזה ראשונה שיהיה יחס כל שלשה מספרים בשורה אחת יחס מתדבק | ||||||||||||||||||||||||||||||||||||||||||||||||||
| The required ratios are produced from them. | ויתילדו מהם מהיחסים הדרושים | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
ראשונה יחסי הכפולים | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונמצא מה שבבית השני על יחסי השני דמיונים | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומה שבבית השלישי על יחסי השלשה כפלים | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומה שבבית הרביעי על יחסי הארבעה כפלים | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
וילך זה בהתמדה עד אין תכלית | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
וקרה שהיה מספר מה [שבבית השלישי על יחס המוסיף חצי למה] שבבית השני | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומה שבבית הרביעי על יחס המוסיף שליש למה שבבית השלישי | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועל הדרך הזה | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומה שבבית השני מהשורה השנית על יחס ארבעה כפלים למה שבבית הראשון[33] | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומה שבבית השלישי על יחס שני דמיונים ורביע למה שבבית השני | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומה שבבית הרביעי על יחס דמיון ושבעה תשיעיות למה שבבית השלישי | ||||||||||||||||||||||||||||||||||||||||||||||||||
| ? | לא יהיה זה סדור | ||||||||||||||||||||||||||||||||||||||||||||||||||
| When wanting to add to the diagram other ratios instead of the diagram of the multiple ratios: replacing the third column with the first and the first with the third, so the middle remains in its place. | וכאשר נרצה להוסיף לציור היחסים האחרים תמורת ציורנו ליחסי הכפלים נהפוך השורה השנית[34] באורך עד שיפול הראשון השלישי בראשון והראשון בשלישי וישאר האמצעי על ענינו | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר נקח לחבר החבור הנזכר מזה המקום בשנקח הראשון אח"כ נעתיקנו ראשונה בשורה השלישית ויהיה ארבעה | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
אח"כ נחבר הראשון והשני ונעתיק אותו אל השורה השלישית ויהיה ששה | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
אח"כ נחבר הראשון והוא ארבעה והשלישי והוא אחד וכפל השני והוא ארבעה ונעתיק אותו אל הבית השלישי והנה יהיה תשעה | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
וימשכו מספרי השורה על יחס המוסיף חצי וכבר התילדו מיחס הכפל והם הנקראים יחד על שם השנים | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר נעשה זה המעשה בשורה אשר ברחב אשר ליחס השלשה כפלים יצאו לך שלשה מספרים על יחס המוסיף שליש כי שניהם נקראים בשם השלשה | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן הענין בטור הרביעי כי הוא יוציא יחסי המוסיף רביעית | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר תהפך הנחת מספרי המוסיף חצי יולד לך יחס הכפל וחצי | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומהמוסיף שליש יחס הכפל ושליש | ||||||||||||||||||||||||||||||||||||||||||||||||||
| When reversing the numbers of the superparticular ratio and applying the known procedure, the rest of the ratios are produced, and they never cease to be produced from each other endlessly, until the production of all of them from equality is revealed. | וכאשר תהפך מספרי המוסיף חלק ותנהג המנהג הידוע יצאו לך שאר היחסים ולא יסורו מלצאת לך קצתם מקצת אל בלתי תכלית עד שיתגלה התילדות כל אלו מיחס השווי | ||||||||||||||||||||||||||||||||||||||||||||||||||
Reducing the Ratios to Equality |
|||||||||||||||||||||||||||||||||||||||||||||||||||
| One can reverse it and find that all the other ratios are reduced to equality. | ובידך שתהפך ותמצא שאר היחסים כלם ישובו אל יחס השווי | ||||||||||||||||||||||||||||||||||||||||||||||||||
The procedure:
|
המשל בזה שאתה כאשר תניח מספרים ג' על יחס נמשך | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותשמור הקטן בעניינו | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
אח"כ נגרע אותו מן האמצעי ותשים מה שנשאר גבול אמצעי | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
אח"כ תשליך אותו מהגדול כמו הקטן ודמיון כפל השני האמצעי ותשים מה שנשאר גבול שלישי | ||||||||||||||||||||||||||||||||||||||||||||||||||
| One finds a continuous ratio. | תמצא יחס מתדבק | ||||||||||||||||||||||||||||||||||||||||||||||||||
| Apply this procedure on these numbers and terms and another ratio will be produced. | אחר כן תעשה באלה המספרים והגבולים זה הפעל ויצא לך יחס אחר | ||||||||||||||||||||||||||||||||||||||||||||||||||
| According to this way until reaching the equality. | ועל הדרך הזה עד שיביא אותך אל יחס השווי | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה שיהיו המספרים ראשונה על יחס שני דמיונים ושתי שלישיות כמו ט' וכ"ד וס"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
|
והנה תשמור ט' | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
|
ותפיל אותם מכ"ד ותשים מה שנשאר והוא ט"ו גבול שני | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
|
והנה תקח כפלו עם ט' ותפיל אותם מס"ד וישארו לך כ"ה והנה תשים אותם שלישי | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
יצאו לך מספרים נמשכים על יחס המוסיף שני שלישים | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
אח"כ תעשה זה המעשה במה שאצלך ויצאו לך ט' וו' וד' והם מספרים נמשכים על יחס המוסיף חצי | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
אח"כ תעשה זה המעשה באלה המספרים ויצאו לד' ב' א' וזה על יחס הכפל | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
אח"כ כשתעשה זה המעשה יצאו לך א' וא' ואחד וחזר אל יחס השווי | ||||||||||||||||||||||||||||||||||||||||||||||||||
| According to this property, when setting the triple ratio, the quadruple ratio and the other ratios that are not mentioned - reducing them to equality by reversing the method by which they were produced. | ובזה הוא הענין כאשר תשים יחס הג' כפלים והד' כפלים ושאר היחסים אשר לא נזכרו התכה בהפך להשיב אותם על יחס השווי מהדרך אשר ממנו הרכבו | ||||||||||||||||||||||||||||||||||||||||||||||||||
Composing a Ratio from Two Ratios |
|||||||||||||||||||||||||||||||||||||||||||||||||||
| Moving on to the composition of a ratio from two ratios. | ונעתק עתה בחבור יחס במספרים משני יחסים | ||||||||||||||||||||||||||||||||||||||||||||||||||
| Prefacing a general introduction enough for examining the property in each ratio, which is that every particular example brought for the composition of a ratio from two ratios, the ratios are already found in this section by a certain description and this rule is possible for all numbers in these ratios. | ונקדים לזה הקדמה כוללת יספיק לנו ממנה בחינת הענין ביחס יחס והיא שכל משל חלקי נביא לחבור יחס במספרים משני יחסים הנה כבר נמצא היחסים בזה החלק על תאר מה והנה זה כלל עובר בכל מספרים יהיו על אלו היחסים | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
ויהיה א"ב על דרך משל ד' ויהיה א"ג ב' ויהיה א"ד ג' | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
ויהיה לא"ב אל א"ד יחס והוא יחס המוסיף שליש | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
ויהיה לא"ד אל א"ג יחס והוא יחס המוסיף חצי | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולא"ב אל א"ג יחס והוא יחס הכפל והוא מחבר בלי ספק משני אלו היחסים | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה אומר שכל יחס המוסיף חצי יצטרף אליו יחס המוסיף שליש יהיה המקובץ מה שתתקבץ הנה בעינו וכל יחס הכפל הנה יסבול שיחלק לאלו השני יחסים ויפרד אליהם | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
שאם לא | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה יהיה יחס ה"ח לה"ז יחס המוסיף חצי | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
ויחס ה"ו לה"ח יחס המוסיף שליש | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה אומר שיחס ה"ו לה"ז יחס הכפל | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
כי אתה יודיע שכאשר נחלק הנה ירצה הבדלנו יחס ב"ד ו"ח אל א"ד ה"ח א' | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
ובהבדל יחס א"ג ה"ז אל ג"ד ז"ח א' | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה בשווי יחס ב"ד ד"ג כמו יחס ו"ח ח"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
ויהיה בהרכבה יחס כל ב"ג אל ד"ג וכל ו"ז אל ח"ז א' | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמנם יחס ד"ג ג"א כמו ח"ז ז"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה בדרך השווי יחס ב"ג ג"א כיחס ו"ז ז"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה בדרך ההרחבה יחס א"ב א"ג הוא יחס ה"ו וז"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||
| Also for the supposition of the compound ratio, it is as it is for this part or for whichever ratios. | וגם כן כאשר תהיה ההנחה היחס המורכבת הנה הוא כאשר היה בזה החלק וביחסים כמו שהיה | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
אח"כ נביא איזה שני מספרים שיהיו | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה יהיו ה"ז ה"ו ויהיו על יחס הכפל | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה נאמר שיחס המוסיף חצי יפול בין ז' וח' | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
שאם לא יפול מחוץ כמו ז' ט' | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה כאשר נצרף אליהם היחס האחר כמו ט"ו ישוב היחס המורכב הראשון | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
ויהיה אז יחס ה"ו ה"ז כמו יחס ה"ו ה"ח כפי מה שבארנו | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
והיה מה שהוא יותר גדול מן ה"ו כמו ה"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה א"כ יפול מבפנים כמו ח' | ||||||||||||||||||||||||||||||||||||||||||||||||||
| ? | ונאמר שיחס ה"ו ה"ח הוא היחס האחר שאם לא הנה יפול ה"ח עם ה"ב או ה"ח או עם ה"ט ויקרה הבטל הנזכר | ||||||||||||||||||||||||||||||||||||||||||||||||||
| We think that we brought a partial proof, as we have mentioned the sesquialter, the sesquitertian and the double ratio, but you should know that this is a general proof. | והלא נחשוב שאנחנו הבאנו מופת חלקי לזכרנו החצי והשליש ויחס הכפל אבל ראוי שתדע שזה המופת כללי | ||||||||||||||||||||||||||||||||||||||||||||||||||
| We brought the example to give the meaning, else you can say that the two numbers AB and AG are two numbers having a certain ratio between them. | ואמנם הבאנו משל לתת המובן שאם לא הנה לך שתאמר ששני מספרי א"ב א"ג שני מספרים חלקים וביניהם יחס מה | ||||||||||||||||||||||||||||||||||||||||||||||||||
| Thus, in this example two ratios AB : AD and AD : AG, were joined into whichever ratio it will be. | וככה חוברנו בזה המשל משני יחסים א"ב א"ד וא"ד א"ג איזה יחס היה | ||||||||||||||||||||||||||||||||||||||||||||||||||
| ? | והנה אם נפל מספר ביניהם קטן מאחד מהם וגדול מאחר אח"כ במופת על הצד הכולל מזולת רמז אל שינוי היחס | ||||||||||||||||||||||||||||||||||||||||||||||||||
| This explanation is enough for endeavoring to confirm the proof of the composition of a ratio from two ratios by numbers as found in the teaching of music after this section. | הנה זה הביאור יספיק ממנו ההשתדלות בקיום המופת על חבור יחס משני יחסים במספרים כאשר נמצא המשלים יוציאו אליו השני יחסים בלמודינו המוסיקי אחר זה האופן | ||||||||||||||||||||||||||||||||||||||||||||||||||
| But, we will endeavor in special explanations [to demonstrate] which ratios are as principals for the other ratios. | ואבל אנחנו נשתדל בבאורים מיוחדים ליחס מה הם כמו ראשים לשאר היחסים | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
מזה שאנחנו נאמר שיחס הכפל ויחס המוסיף חצי יחובר מהם יחס הג' דימיונים א"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
מופת זה | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
שא"ג יכפל א"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה ב"ג כמו א"ב הנה הוא חצי א"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמנם ג"ד חצי א"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה א"ב ב"ג ג"ד שוים קצתם לקצת | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה יהיה כל א"ד ג' דמיוני א"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם יהיה ג"ד שליש א"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה א"ד כפל ושליש א"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה נחלק א"ג אל שלשה חלקים על ה' וז' | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה יהיה א"ה כמו ג"ד והוא שליש א"ג אשר הוא כפל א"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה חצי א"ה שליש א"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה א"ד כמו כפל א"ב רצו' א"ג וכמו שלישית רצו' ג"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם היה יחס א"ג א"ב יחס המוסיף שליש | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה יחס א"ד א"ב יחס הכפל | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה נחלק א"ב בשני חלקים על ה' | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
ויהיה א"ה כמו ב"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
ויהיו חלקי א"ה ה"ב ב"ג שוים והם ג' | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
וג"ד כמו א' מג' חלקי א"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה החלקים הד' שוים | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה כלל ב"ד כמו כלל א"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה תוספת א"ד על א"ב בדמיון | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם היה יחס א"ג א"ב יחס המוסיף שליש | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
ויחס א"ד א"ג יחס המוסיף שמינית | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה יחס א"ד א"ב יחס המוסיף חצי | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה נחלק א"ב לשלישיותיו על ז' וה' | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
ויהיו חלקי א"ז ז"ה ה"ב ב"ג שוים והם ד' חלקים | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
וחצי כל אחד מהם הוא שמינית א"ג והוא שוה לג"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה יהיה ב"ד שלשה דמיוני ג"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
וא"ב ששה דמיוני ג"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
והוא יחס דמיון וחצי | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
ויחס ב"ד ד"ג הוא יחס א"ב ב"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
ובתמורה יחס ב"ד א"ב יחס ד"ג ב"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
וד"ג חצי ב"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה ב"ד חצי א"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה א"ד א"ב יחס דמיון וחצי | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר היה יחס א"ג א"ב יחס דמיון ורביע | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
ויחס א"ד א"ג דמיון וחומש | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה יחס א"ד א"ב יחד דמיון וחצי | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
לפי שא"ב כאשר יתחלק ברביעיות היה כל חלק כמו ב"ג והיו החלקים חמשה שוים ויהיה ב"ד כמו חצי א"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר היה יחס א"ג א"ב יחס דמיון וחומש | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
ויחס א"ד א"ג יחס דמיון וששית | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה יחס א"ד א"ב יחס דמיון ושתי חמשיות | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
ויתבאר לך זה כשנחלק א"ב לה' חלקים ונעשה מה שעשינו | ||||||||||||||||||||||||||||||||||||||||||||||||||
Therefore, it is clear that:
|
ויתבאר לך מזה שיחס המחובר מדמיון וששית ודמיון ושביעית הוא יחס דמיון ושליש | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
והמחובר מדמיון ושביעית ודמיון והשמינית הוא יחס דמיון ושתי שביעיות | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
והמחובר מדמיון ושמינית ודמיון ותשיעית הוא יחס דמיון ורביעית | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
והמחובר מיחס דמיון ותשיעית ודמיון ועשירית הוא יחס דמיון ושתי תשיעיות | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
והמחובר מדמיון ועשירית ודמיון וחלק מי"א הוא יחס דמיון וחומש | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
והמחובר מדמיון וחלק מי"א ודמיון וחלק מי"ב הוא יחס שני חלקים מי"א | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
והמחובר מדמיון וחלק מי"ב ודמיון וחלק מי"ג הוא יחס הששית | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
והמחובר מדמיון וחלק מי"ג ודמיון וחלק מי"ד הוא יחס משני חלקים מי"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
והמחובר מיחס הדמיון וחלק מי"ד ודמיון וחלק מט"ו הוא יחס דמיון ושביעית | ||||||||||||||||||||||||||||||||||||||||||||||||||
| And so on continually. | ועל הדרך הזה כל ההמשך | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר היו א"ג א"ב על יחס דמיון וחלק מט"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
וא"ד א"ג על יחס המוסיף רביעית | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה יחס א"ד א"ב דמיון ושליש | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה לפי שאתה כאשר תחלק א"ב בט"ו חלקים | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
יהיה כל א"ג י"ו חלקים | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
וג"ד רביעית זה הנה הוא ד' חלקים | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה כל ב"ד ה' חלקים | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
וא"ב [ט]"ו חלקים | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכל א"ד כ' חלקים | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה ב"ד שליש א"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכמו זאת ההנהגה הנה הוא כאשר היה א"ג א"ב על יחס המוסיף חצי | ||||||||||||||||||||||||||||||||||||||||||||||||||
| When following this way it is possible to bring a proof for the rest of the composition in music, as the previous explanation is enough for the endeavoring in all this. | ואפשר לך כאשר תלך בזה הדרך שתביא מופת על שאר מה שבמוסיקי מהחבור לפי שהביאור הקודם יספיק לך השתדלות בזה כלו | ||||||||||||||||||||||||||||||||||||||||||||||||||
| The third section is complete. | נשלם המאמר השלישי' | ||||||||||||||||||||||||||||||||||||||||||||||||||
Numbers as Geometric Shapes |
|
| The properties of the number from the aspect of its quantity by itself were already referred to. | כבר רמזנו לך אל ענייני המספר מצד כמותו בעצמו |
| [Now] the properties of the number from the aspect of its having a quality of combination from units, by which it resembles to the measured shapes, will be referred to. | ורמזנו לך אל עניינים מענייני המספר מצד שיש לו איכות חבור מהאחדיות ידמה בו לתמונות השעוריות |
| The images of the number resemble by combinations to the measures. | כבר ידמו תמונות המספר בחבורים לשעורים |
[They are called]:
|
ויאמר מספרים קוויים |
|
ומספרים שטחיים ומושטחים |
|
ומספרים גשמיים ומוגשמים |
Linear Numbers |
|
| The linear numbers begin from one and follow their natural course. | הנה המספרים הקוויים מתחילים הם מהאחד וילכו כפי מנהגם |
| The first linear number is two, then three and so on. | והמספר הקוי הראשון הוא השנים אח"כ הג' ועל הדרך הזה |
Plane Numbers |
|
| The plane [numbers] are those that can be combined to one another by a combination that resembles to the one that have a geometrical shape. | ואולם המושטחי' הנה הם אשר אפשר שיחובר קצתם אל קצת חבור ידמה קצת השטים הבעל תמונה |
| והמוגשמים | |
Triangular Numbers |
|
| The first plane [numbers] are the triangular numbers. | והמושטחים ראשונים הם המספרים המשלשים |
| They are the numbers that when their units are arranged in a certain arrangement they resemble to a geometrical shape that is encompassed by three sides. | והם המספרים אשר כשיסודרו אחדיהם סדור מה ידמו תמונה יקיפו בה שלש צלעות |
|
והראשון שבהם שלשה וצורתם כזה |
|
אח"כ הששה |
| Their shape is generated from joining a numerical line that exceeds by one over the numerical line [of the preceding triangle] | וצורתם תתחדש מצרוף קו מספריי מוסיף באחד על הקו המספריי אשר הוא כמו שראית |
|
שנים נצטרף אל האחד ונתילד המשלש הראשון והנה יהיה שלשה ותהיה הצורה בזה |
| According to this way, whenever a numerical line is joined, by the sequence of the numbers, a greater triangle will generated. | ועל הדרך הזה כל מה שתצטרף אל זה קו מספריי על סדר המספרים הנמשכים יתחדש משלש יותר גדול |
|
המשל בזה כשתצרף אל זה קו מספריי מארבעה אחדויות יהיה תמונה משלש אחר כזה |
|
והנה הראשון שבמשלשים ג' וצלעו ב' |
|
והמשלש השני ששה וצלעו ג' |
|
והמשלש השלישי י' וצלעו ד' |
|
והמשלש הרביעי ט"ו וצלעו ה' |
|
והנה כל משלש יוסיף על אשר ימשך תחתיו כמו צלע עצמו |
|
ויתחלפו צלעותיהם על סדר המספרים הנמשכים מן האחד עם הא' ואי זה מספר שיתקבץ מזה הנה הוא משלש |
|
וכל משלש הנה צלעו יוסיף על מדרגתו בא' |
|
וכאשר יאמר לך מה הוא צלע המשלש העשירי מראשית המספרים המשלשים |
|
יהיה מספר הצלע ומספר המדרגה אחד |
|
ואם יאמר אליך שתאמר שהוא מרבע או מעקב בכח הנה אינו משלש ולא מחומש ולא דבר מאלו לא בכח ולא בפועל אלא בשתוף השם ולא תשגיח למה שיאמרו |
|
וכל משלש הנה הוא חצי הכאת מדרגתו במוסיף ממנו באחד |
|
עד שאם יאמר לך מהו מספר המשלש החמשי בכח הנה תקח חמשה ותכה אותם במוסיף ממנו באחד ויהיו שלשים ותקח חציים והוא חמשה עשר והוא המשלש החמישי[35] |
|
וכל צלע משלש הנה הוא היותר קטן שבשני מספרים נמשכים יוכה אחד מהם באחר ויהיה ממנו כפל משלשו |
|
עד שאם יאמר לך מה הוא צלע חמשה עשר מהמשלשים |
|
הנה אנחנו נכפול אותו ויהיה שלשים ונבקש שני מספרים נמשכים אשר מושטחם שלשים ונמצא שהם חמשה וששה ונאמר שצלעו חמשה |
Square Numbers |
|
| After the triangular numbers are the square numbers. | ואחר המספרים המשלשים המספרים המרבעים |
| They are as already known. | והם אשר כבר ידעת אותם |
| They are generated from the numerical lines, the value of each of which is equal to the number of units in it. | והנה הם יתחדשו מקוים מספריים שוים מספרם מספר מה שבאחד מהם מן האחדים |
| Their sides are according to the order of the numbers, beginning from one. | וצלעותיהם על סדר המספרים מתחילים מן האחד |
|
כמו האחד שהוא מרבע האחד |
|
|
והארבעה שהוא מרבע השנים |
|
|
והתשעה שהוא מרבע השלשה |
|
|
והששה עשר שהוא מרבע הארבעה |
|
כפי הצורות האלו |
|
והתילדותם מקבוץ הנפרדים הנמשכים עם האחד |
|
כמו השלשה והאחד שהוא ארבעה והוא ראשון המספרים המרבעים[36] |
|
אח"כ האחד והשלשה והחמשה והוא תשעה והוא המספר המרבע השני |
|
אח"כ האחד והשלשה והחמשה והשבעה והוא ששה עשר והוא המספר המרבע השלישי |
|
ומסגלות המרבעים שאתה כאשר תקבץ אותם ממרבע האחד יהיה מקובצם יותר ממרבע האחרון כמו מה שלפניו מן המרבעים |
|
המשל בזה שמקבץ מרבע האחד והשנים יוסיף על מרבע השנים כמו מרבע האחד |
|
ומקובץ מרבעי האחד והשנים והשלשה יוסיף על מרבע השלשה כמו מקבץ שני מרבעי האחד והשנים |
|
וכבר הוציאו להולדת המרבעים דרך יקראוהו רצוא ושוב[37] |
|
והוא שאתה כאשר תתחיל מן האחד ותקבץ מה שתרצה מהמדרגות אח"כ תהיה נוטה יורד ומקבץ הנה מה שיהיה מקובץ זה ההוא מרבע |
|
המשל בזה שיעלה מן האחד אל השנים ויהיו שלשה אח"כ נשוב אל האחד ויהיו ארבעה והוא מרבע ראשון |
|
אחר כן כאשר תקבץ האחד והשנים והשלשה ותצרף אליו השנים והאחד יהיו תשעה והוא מרבע שני |
|
וכאשר תעלה מן האחד והשנים והשלשה והארבעה מקובץ אח"כ תרד ותקבץ השלשה והשנים והאחד יהיה מקובץ זה ששה עשר והוא המרבע השלישי מן המרבעים המספריים |
|
ושלמות זה הדרך שמקובץ כל המספרים הנמשכים עם מקובץ מה שהוא חסר מהם כמו המדרגה האחרונה הנה הוא מרבע |
|
וגם כן כפל מקובץ כל מספרים נמשכים זולת המספר האחרון הנה הוא מרבע |
|
וכל שני משלשים נמשכים יתקבצו מן האחד והשלשה והששה הנה הוא מרבע וזה גם כן התילדות המרבעים |
|
ויהיה כל מרבע ממשלש במדרגתו ומשלש חסר ממדרגתו באחד |
|
וכל שני מרבעים יוכה צלע אחד מהם באחר ויכפל ויקובץ אל השני מרבעים הנה הכל מרבע[38] |
|
כמו הכאת שנים בשלשה כאשר יקובץ כפלו עם ארבעה ותשעה אשר הוא חמשה ועשרים |
|
וכל מרבע יתוסף עליו גדרו פעמים ואחד או יצורף אל דמיונו ודמיון רביעיתו או שלשה דמיוניו או יחוסר ממנו שלשה רביעיותיו הנה מה שיגיע מרבע[39] |
|
ואין מרבע שיהיה חציו או כפלו מרבע |
|
ולא יתקבצו מהמרבעים הנמשכים המתחילים מן האחד מרבע כלל |
| ? | ואין מרבע שיהיה לו שליש מן השלמים |
|
ודע שאחדי המספר הנגדר לא ימלטו אם שיהיו אחד או ארבעה או חמשה או ששה או תשעה |
|
ואם היה אחד הנה אחדי צלעו אם אחד ואם תשעה |
|
ואם היה ארבעה הנה הם שנים או שמנה |
|
ואם היה חמשה הנה הם חמשה |
|
ואם היה ששה הנה הם ארבעה או ששה |
|
ואם היה תשעה הנה הם שלשה או שבעה[40] |
|
ובחינת[41] המרבעים בדרך אנשי הודו הנה לא ימלט אם שיהיה אחד או ארבעה או שבעה או תשעה |
|
והנה לאחד אחד או שמנה |
|
ולארבעה שנים או שבעה |
|
ולשבעה ארבעה או חמשה |
|
ולתשעה שלשה או ששה או תשעה |
Polygonal Numbers |
|
| Following the square numbers are the pentagonal numbers | וימשכו למספרים המרבעים המספרים המחמשים |
|
והראשון שבהם החמשה ויתחברו כזה |
|
והוא המחמש הראשון וצלעו שנים |
|
והמחמש השני הוא אשר צלעו המספר השני והוא שלשה ויהיה המחמש המתקבץ ממנו שנים עשר כזה |
|
והמספר השלישי והוא ארבעה הנה המחומש המתקבץ ממנו הוא שנים ועשרים כזה |
| Likewise the fourth, the fifth, the sixth, and the seventh - the sequence of their sides is as the sequence of the successive numbers. | והרביעי והחמשי והששי והשביעי סדור צלעותיהם על סדור המספרים הנמשכים |
|
והתילדותם מקבוץ המספרים העודפים בשלשה שלשה מתחילים מן האחד |
|
כמו מספרי א' ד' ז' י' י"ג י"ו י"ט כ"ב עם האחד |
|
כי האחד עם הארבעה חמשה והוא המחמש הראשון |
|
והאחד עם הארבעה והשבעה שנים עשר והוא המחמש השני |
|
והאחד עם הארבעה והשבעה והעשרה שנים ועשרים והנה זה המחמש השלישי |
|
וכבר יולדו מקבוץ המרבעים עם המשלשים רצוני קבוץ המרבע אשר במדרגתו עם המשלש אשר הוא למטה ממדרגתו |
|
כמו שתמצא כי מקבוץ המרבע השני שהוא תשעה עם המשלש הראשון שהוא שלשה יולד שנים עשר המחמש השני |
| Indeed, one finds such property in all the measured shapes: | והנה לכל התמונות השעוריות תמצא סגלה כזאת |
|
כי הנה המשושה יתחדש מקבוץ המחמש אשר במדרגתו עם המשלש אשר הוא למטה ממנו במדרגה |
|
כמו המששה השני שהוא חמשה עשר אשר נולד משנים עשר המחמש השני עם שלשה המשלש הראשון |
|
וכן המשבע השני שהוא שמנה עשר יתחדש מהמששה השני שהוא חמשה עשר ומשלשה המשלש הראשון |
|
ועל הדרך הזה |
|
וכבר יולדו המחמשים בלקיחת המרבעים אשר במדרגתם וכפי מספר המדרגות כל כך פעמים נוסיף על המרבע ההוא מספר חצי צלעו |
|
דמיון זה לקחנו ארבעה המרבע הראשון והוספנו עליו חצי צלעו ועלה חמשה המחמש הראשון |
|
ולקחנו תשעה המרבע השני וקבצנו עמו שני פעמים חצי צלעו ועלה שנים עשר המחמש השני |
|
ולקחנו ששה עשר המרבע השלישי והוספנו עליו שלשה פעמים חצי צלעו ועלה שנים ועשרים המחמש השלישי |
| This property is also related to all the measured shapes: | וכן הסגלה הזאת דבקה לכל התמונות השעוריות |
|
אמנם למשושה תמורת חצי צלע נקח צלע |
|
ולמשבע תמורת זה צלע וחצי |
|
ולמשמן שתי צלעות |
| So on, adding half the side by half the side, then multiplying the result by the number of the ranks. | וכן תמיד בתוספת חצי צלע חצי צלע אח"כ בהכפלת המגיע במספר המדרגות |
|
משל זה לקחנו ארבעה המרבע הראשון וקבצנו עמו צלעו פעם ועלה ששה המששה הראשון |
|
לקחנו תשעה המרבע השני וקבצנו עמו צלעו שתי פעמים ועלה חמשה עשר המששה השני |
|
עוד לקחנו ארבע המרבע הראשון וקבצנו עמו צלעו וחצי צלעו פעם ועלה שבעה המשבע הראשון |
|
לקחנו תשעה המרבע השני ועמו קבצנו שני פעמים צלעו וחצי צלעו ועלה שנים עשר המשבע השני ועל הדרך הזה כלם |
| All these are composed from triangles. | וכבר התחברו אלה כלם מהמשלשי' |
|
וכמו שהמרבע יתרכב משני משלשים |
|
כמו כן המחמש יתרכב משלשה |
|
והמששה מארבעה |
|
והמשבע מחמשה |
| In a way that is similar to the composition of the squares. | על דרך דומה לדרך חבור המרבעים |
|
ויהיה דרך משל המחמש השני שהוא י"ב מרכב מהמשלש הראשון שתי פעמים והמשלש השני פעם |
|
והמחמש השלישי מהמשלש השני שתי פעמים והמשלש השלישי פעם |
|
וכן המששה השני שהוא ט"ו מורכב מהמחמש השני המורכב משלשה משלשי' ומהמשלש הראשון אשר למטה ממנו |
|
ואפשר לומר לפי זה שיהיה האחד ג"כ כן במדרגת משלש |
|
וזה כי המחמש הראשון הוא מורכב מארבעה ואחד |
|
וכל מרבע שעוריי יתחלק לשני משלשים ולזה מן הנכון שיהיה האחד כמו כן במקום משלש אחד |
|
וכל מששה משלש ולא יתהפך |
|
כי הנה הששה מששה ראשון הוא המשלש השני |
|
וט"ו המששה השני הוא המשלש הרביעי |
|
וכ"ח המששה השלישי הוא המשלש הששי |
|
ואין כל משלש מששה |
|
כמו ג' י' וכ"א שהם משלשים ואינם מששים |
|
וכל משלש מספרו זוג הנה אין שתוף בינו ובין המששה[42] |
|
וכאשר תרצה שתמצא מדרגת המשלש מהמששה הנה תגרע האחד מכפל מספר מדרגת המששה[43] והפוכו שתוסיף אחד על מספר מדרגת המשלש ותקח חציו |
|
וכל מספר מחמש הנה הוא חצי מה שיתקבץ מהכאת מספר חסר ממדרגתו באחד בתוספת אשר בין המספרים אשר יתילדו מהם[44] והוא שלשה נוסף עליו מה שבין שני מספרים מזה והוא שנים מוכה במספר מדרגתו מהמחמשים המספריים |
|
המשל בזה כאשר תרצה שתדע המחמש הרביעי תכה שלשה בשלשה ויהיו תשעה ותוסיף עליהם שנים ויהיו אחד עשר תכה אותם בארבעה ויהיו ארבעים וארבעה תקח חצים ויהיו שנים ועשרים והוא המחמש הרביעי |
|
וגם כן כי כל מחמש הנה הוא כמו הכאת מספר מדרגתו נמנית מן האחד בעצמו נוסף עליו חצי צלעו בתוספת מדרגתו במחמשים המספריים[45] |
|
המשל בזה בשאלה הנזכרת שנכה ארבעה בארבעה לפי שהוא במדרגה הרביעית מן האחד ויהיו ששה עשר ותוסיף עליו חצי צלעו והוא שנים ושלשה מדרגות ויהיו שנים ועשרים[46] |
| After the pentagons the hexagons | ואחר המחמשים המששים |
|
ויתחברו מקבוץ המספרים העודפים בארבעה ארבעה |
|
כמו א' ה' ט' י"ג י"ז כ"א |
|
על הקש מה שנאמר במחמשים |
| Then the heptagons | אח"כ המשבעים |
|
ויתחברו מקבוץ המספרים העודפים בחמשה חמשה |
| The same is applied to all of them. | וכן תקיש בכלם |
|
ונאמר שכל שטח אחר המשלש כאשר יחובר עם המשלש יתחדש השטח אשר ימשך לזה השטח במספר הצלעות |
|
כמו המשלש הראשון והוא שלשה כשיתחבר עם המרבע השני יהיה מחמש |
|
וכאשר יתחבר עם המחמש השני והוא שנים עשר יהיה מששה והוא החמשה עשר |
|
ועל זה הדרך |
|
ומותר כל שטח על אשר לפניו |
| ? | וכבר נזדמן זה ולא יתהפך |
|
וכל מספר שלם הנה הוא לפניו מששה או משלש |
|
ועוד יהיה מזה דרך נגיע בו אל הוצאת מדרגות המספרים השלמים גם כן |
|
כי כאשר יאמר לך המספר השלם הראשון מאיזה מהמשלשים או מהמששים הוא |
|
הנה תעיין אל הסדור אשר ידעת אותו בזה האחד בפרט |
|
ותמצא הראשון שבזוגי הזוגות יבחן בו הסדור הידוע ארבעה |
|
וכאשר תגרע ממנו אחד ישאר שלשה מספר ראשון ונכון הוא לפעלתך |
|
|
ותקח חציו והוא שנים |
|
ותאמר שהוא המששה השני והמשלש השלישי |
|
וימשך אל הארבעה שמנה |
|
ותמצא השבעה כמו זה מספר ראשון ויתכן למבוקשך |
|
|
ותחלק לחציים השמנה ויהיה ארבעה |
|
ותאמר שהוא המששה הרביעי והמשלש השביעי |
|
וימשך לשמנה ששה עשר |
|
וכאשר תגרע ממנו אחד ישאר מורכב והנה איננו נכון לפעלתך |
|
וימשך אל הששה עשר שנים ושלשים |
|
וכאשר תגרע ממנו אחד ישאר ראשון והוא נכון לפעלתך |
|
|
ותקח חציו והוא ששה עשר |
|
והנה תאמר המששה הששה עשר והמשלש האחד ושלשים |
|
ועל זה ההקש |
| See the wonderful diagram, from which it is clear that all the geometric shapes are produced from the triangle and dissolved to it: | ראה צורה נפלאה יתבאר ממנה שכל התמונות מתילדי' מהמשלש ונתכים אליו |
| מעשרים | מתשעים | משמנים | משבעים | מששים | מחמשים | מרבעים | משלשים |
| י | ט | ח | ז | ו | ה | ד | ג |
| כז | כד | כא | יח | טו | יב | ט | ו |
| נב | מו | מ | לד | כח | כב | יו | י |
| פה | עה | סה | נה | מה | לה | כה | טו |
| קכו | קיא | צו | פא | סו | נא | לו | כא |
| קעה | קנד | קלג | קיב | צא | ע | מט | כח |
| רלב | רד | קעו | קמח | קכ | צב | סד | לו |
| רצז | רסא | רכה | קפט | קנג | קיז | פא | מה |
| שע | שכה | רפ | רלה | קצ | קמה | ק | נה |
| decagon | nonagon | octagon | heptagon | hexagon | pentagon | square | triangle |
| 10 | 9 | 8 | 7 | 6 | 5 | 4 | 3 |
| 27 | 24 | 21 | 18 | 15 | 12 | 9 | 6 |
| 52 | 46 | 40 | 34 | 28 | 22 | 16 | 10 |
| 85 | 75 | 65 | 54 | 45 | 35 | 25 | 15 |
| 126 | 111 | 96 | 81 | 66 | 51 | 36 | 21 |
| 175 | 154 | 133 | 112 | 91 | 70 | 49 | 28 |
| 232 | 204 | 176 | 148 | 120 | 92 | 64 | 36 |
| 297 | 261 | 225 | 189 | 153 | 117 | 81 | 45 |
| 370 | 325 | 280 | 235 | 190 | 145 | 100 | 55 |
|
וזה שאתה כשהתחלת מן האחד ראשון המשלשים בכח וצרפת אותו אל השלשה ראשון המשלשים בפעל נתילד מהם ארבעה ראשון המרבעים בפעל |
|
וכאשר צרפת ג' עם ו' הנמשך לו נתילד מהם ט' המרבע השני |
|
וכן תמיד |
|
אח"כ כשתשב אל האחד ראשון המשלשי' בכח וצרפתהו עם ד' ישוב ה' מחמש ראשון בפעל |
|
ובצרוף ג' לט' יתילד י"ב מחמש שני |
|
ומו' וי"ו מחמש שלישי |
|
וכשתשוב לאחד ותצרפהו עם ה' יוליד מששה ראשון בפעל |
|
וג' עם י"ב יוליד מששה שני |
|
וכן תמיד |
Solid Numbers |
|
| Now the solid numbers will be discussed. | ונדבר עתה במספרים המוגשמים |
Pyramids with Sharp Apex |
|
| The first of them are the pyramids. | והראשונים שבהם המחדדים |
| The pyramids are the most famous of the sharp solids, beginning from a broad base, then they do not cease from growing, until reaching the end of the sharp limit, the one. | והמחדדים והם הנודעים במתלהבים הם אשר יתחילו מתושבת מרוחת אח"כ לא יסורו מלצמוח עד שיגיעו אל קצה הגבול מן החדות אל האחד |
|
והראשון שבהם אשר תושבתו משלשת |
|
והראשון מאלו הם הארבעה והנה הם המספר הראשון אשר הוא קויי ושטחיי ומגשם |
Their production:
|
ויתילד מחבור המשלשים על המשכם |
| ?? | הדרכה לחסרים מהם על המוסיף עד שיכלו אל האחד |
|
אח"כ אשר תושבתם ארבעה ויתילדו ומחבור המרבעים על זה התאר |
|
ועל הדרך הזה אשר תושבתם מחמשת ואשר תושבתם משששת |
| ?? | וכל מספר מושטח יתרכבו ממנו יקרא ואשר יחסר מצדו יקרא |
| Their production: | והתילדותם |
|
אם אשר תושבתו משלש |
|
הנה כאשר יצורף אל האחד המשלש הראשון ויהיו ארבעה והנה הוא המחדד הראשון |
|
וכאשר יצורף עם אלו עוד המשלש השני ויהיו עשרה יעלה המחדד השני מזה המין |
|
ואמנם אשר תושבתם מרבע |
|
הנה הראשון שבהם מן האחד והמרבע הראשון |
|
והשני אליו מן האחד והמרבע הראשון והמרבע השני |
|
ואשר תושבתם מחמש ומששה וזולת זה הנה הם על זה ההקש |
| The property of the angles and the sides and their number is according to the rule of the geometric shapes that have magnitude. | ואמנם ענין הזויות והצלעות ומספרם הנה הוא על הקש התמונות בעלות הגודל |
Prisms |
|
| The prism is also among the numerical solid shapes. | והמתגורר גם כן מהתמונות המספריות המוגשמות |
| It is [produced] from multiplying the triangles and their relation to one another. | והוא מהכפלת המשלשים ודבקות קצתם בקצת |
|
והששה המגורר הראשון שיתילד מן המשלש הראשון לו שלשה צלעות כל צלע בעל ארבעה ושתי צלעות כל צלע משלש |
| ? | אמנם הצלעות במספרם |
| The solid shapes encompassed by six surfaces are necessarily: | ואמנם התמונות המגשמות אשר יקיפו בהם ששה שטחים הנה לא ימלטו |
|
אם שיהיו ארכם ורחבם ועמקם שוים ויהיו כמו עשר בעשר אח"כ בעשר ויקרא מעקב |
|
ואם שיהיו שני קטרים מהם שוים וקטר מתחלף |
|
וכאשר יהיה הקטר המתחלף יותר קטן יקרא לבניי |
|
וכאשר יהיה יותר גדול יקרא עמודיי |
|
אם יהיה מושטחו הקטן עגלה יקרא מעגל |
| ?? | כמו חמשה בחמשה אח"כ ביותר מחמשה |
| באשר היה שיהיו השלשה מתחלפים הנה יקרא כפי זאת הצורה | |
|
ודמיון הלבניי ארבעה בארבעה אח"כ בשלשה |
|
ודמיון העמודיי הארבעה בארבעה אח"כ בחמשה |
| ודמיון שלשה בארבעה בחמשה או בשמנה | |
| ומנהגם שיקראו המספר אשר ישוב כשהוכה בעצמו בעצמו אח"כ מה שנתקבץ כעצמו ועל הדרך הזה מספר עגול כמו בס' החמשה והששה | |
|
|
כי החמשה בעצמו חמשה ועשרים |
|
|
אח"כ בחמשה מאה ועשרים וחמשה |
|
|
והששה בעצמו ששה ושלשים |
|
|
אח"כ בששה מאתים וששה עשר |
| יש מן האנשים מי שיקראו משטחו עגלה ומעוגל ומעקבו כדור וכדוריי |
Cube Numbers |
|
| The properties of the cube that should be investigated and what is are already known in the book of The Elements: | ואשר ראוי שנחקור מעניני המעקב וכבר נודע ממנו כלל בספר היסודות |
|
ומסגלות המעקב שעקב כל מספר כאשר הוכה באשר ימשך אליו אח"כ באשר לפניו אח"כ יתוסף אשר לפניו על מה שיתקבץ יהיה שוה לו[47] |
| Their production is when the successive odds are arranged, beginning from the one, then summed according to the rank, thus the cubes are produced successively. | אמנם התילדותם הנה כאשר יסודרו הנפרדים הנמשכים מתחילים מן האחד ואח"כ יקובצו כפי המדרגה הנה יתילדו המעקבים על המשכם |
|
המשל בזה שיסודרו אחד שלשה חמשה שבעה תשעה אחד עשר שלשה עשר |
|
הנה האחד מעקב |
|
ואחריו השלשה והוא במדרגה השנית הנה יחויב שתקבץ שתי מדרגות ותקבץ השלשה והחמשה והנה זה שמנה והוא מעקב |
|
ואחריהם השבעה והוא במדרגה השלישית והנה יחויב שתקבץ שלשה מדרגות והנה יהיו שבעה תשעה אחד עשר וכלל זה שבעה ועשרים והוא המעקב השלישי |
|
ועל זה המנהג |
| When wishing to know the first odd number, from which a known cube is composed, take the number of the rank of the cube from the first number of the cubes, and it is one less than the number of the terms, | וכאשר תרצה לדעת הנפרד הראשון שנתרכב ממנו המעקב הידוע הנה תקח מספר מדרגת המעקב מהמספר הראשון שבמעקבים והנה יהיה זה חסר מן הראשון באחד |
|
ויהיה דמיון שני אלו במעקב השלישי אם הראשון הנה הוא שלשה ואם השני הנה הוא שנים |
| Subtract the second from the square of the first, | ותגרע השני ממרבע הראשון |
|
כמו שתגרע הנה השנים מתשעה והנה הוא הנפרד הראשון אשר ממנו חבור המעקב השלישי וזה הוא שבעה |
|
אח"כ תוסיף אותו עליו והנה יהיה אחד עשר והוא הנפרד האחרון אשר ממנו הרכבתו |
|
ונתרכב מהם וממה שביניהם[48] |
|
והארבעה והחמשה והששה והתשעה חוזרים במעקביהם תמיד אחדים והנה יהיה זה הוראה על אחדי העקב |
|
כמו ארבעה בארבעה אח"כ בארבעה ויהיו ארבעה וששים |
|
|
והתשעה בתשעה אח"כ בתשעה והוא שבע מאות ותשעה ועשרים |
|
אמנם עקב השנים הנה הוא בשמנה תמיד |
|
ועקב השמנה הנה הוא בשנים תמיד |
|
ועקב השבעה בשלשה |
|
ועקב השלשה בשבעה תמיד |
|
והכאת המעקב במעקב וחלוקתו עליו מעקב |
| ?? | והכאת מרבע שני מספרים במרבע מספר אחד יחסים יחס שני מעקבים |
|
החלוק בין שני מעקבים הנמשכים הנה הוא הכאת היותר קטן שבשני המעקבים במספר אשר ימשך אליו ויוסיף עליו באחד כן בשלשה אחר כן תוסיף עליו אחד[49] |
|
וכל מעקב יוסר ממנו עקבו הנה יהיה לנשאר שתות שלם |
|
וכל מעקב זולת אחד הנה ימנה אותו עקבו זולת אחד |
|
וכל מעקב הנה חציו וכפלו בלתי מעקב |
|
וכל מעקב יחובר אליו האחד והכאת המשלש אשר במדרגתו בששה תמיד הנה הוא המעקב אשר ימשך אליו |
|
והנה אפשר שיתילדו מאלו המעקבים |
|
ומסגלות המעקבים שבחינתם אשר כפי מעשה המספר אשר לאנשי הודו יהיה אם אחד ואם שמנה ואם תשעה |
|
ואם היה אחד הנה אחדי הצלעות אחד או ארבעה או שבעה |
|
ואם היה שמנה הנה הוא שמנה או שנים או חמשה |
|
ואם היה תשעה הנה הוא שלשה או ששה או תשעה |
Heteromecic and square numbers |
|
| The numbers that have sides are divided: | וכבר יתחלקו בעלי הצלעות מהמספר |
|
ויאמר שמהם מה שהוא הוא הוא הארך[50] |
|
ומהם מה שהוא זולתיי הארך |
|
ומהם מה שהוא נבדל הארך והנה הוא אשר ההתחלפות בין ארכו ורחבו במה שהוא למעלה מאחד יהיה איך שיהיה |
| The custom of those who discuss arithmetic to bring in this place a discussion that is derived from the people of demonstration, which is very similar to a rhetorical and poetical discussion, but we reject this and we demonstrate it by calling the heteromecic a heteromecic number. | ומנהג המדברים במלאכת המספר שיביאו בזה המקום ובמה שירוץ מרוצתו דבור יוצא ממנהג אנשי המופת ודומה הרבה למאמר ההלציים והשיריים ונברח מזה ונוכיח עליו בקריאתם הזולתיי הארך בזולתיי הארך |
| It seems that the first "otherness" that falls between the numbers is in one, and it is the basis of the heterogeneity and its beginning, as it is the basis of the number itself. | וידמה שיהיה הזולתיות הראשון שיפול בין המספר והמספר הוא באחד ויהיה הוא שרש ההתחלפות והתחלתו כמו שהוא שרש המספר עצמו |
| The heteromecic numbers are those [whose length and breadth] differ by one. | ויהיו המספרי' הזולתיים הארך הם המשתנים באחד |
| The heteromecic surfaces are those that are encompassed by two heteromecic sides. | והשטחים הזולתיים הם אשר יקיפו בהם שתי צלעות זולתיות |
Setting a table:
|
וכאשר נרשום לוח יסודרו בו הנפרדים על המשכם מתחילים מן האחד בשורה אחת |
|
והזוגות על המשכם מתחילים מן השנים בשורה אחרת |
| As is known, by summing the odd numbers, the square numbers are produced. | הנה יולדו מקבוץ הנפרדים כפי מה שידעתו אותו המספרים המרבעים |
| By summing the even numbers, the heteromecic numbers are produced. | ויולדו מקבוץ הזוגות המספרים הזולתיים הארך |
| The "same" squares are produced from the odd numbers and the "others" from the even numbers as a necessary result. | ויתילדו מן הנפרדים המרבעים ההויים ומן הזוגות הזולתיים כפי החיוב |
| The pythagoreans start from this place, in a way that has no perfection. | ויתחילו הפיתגוריים מזה המקום בדרך אין שלמות לו |
| When summing the squares once again in a line, attributes and properties are seen from the position of the two lines: | וכאשר תקבץ המרבעים פעם שנית בשורה יראה משכונת שתי השורות ענינים וסגלות |
|
ומהם שאתה תמצא ראשון הזולתיים על יחס הכפל מראשון המרבעים והוא המוסיף דמיון |
|
והשני אצל השני על יחס המוסיף חצי |
|
והשלישי אצל השלישי על יחס המוסיף שליש |
|
ועל הדרך הזה כלם על דרך המספרים והמדרגות |
|
המשל בזה שהוא לרביעי רובע |
|
ולחמישי חומש |
| One finds the exceedance according to the ratio of the natural numbers: | ותמצא ההעדף על יחס המספרים הטבעיים |
|
כי הנה העדף המדרגה הראשונה אחד |
|
והעדף המדרגה השנית שנים |
|
ועל הדרך הזה |
| When one is omitted and the numbers are set vis-a-vis, the ratio is like this: | וכאשר ישמט האחד ויהיה הנכחיות בין מה שהוא מספר יבא היחס כמו זה |
| The excess is [now] in what was [previously] deficient: | ואמנם התוספת מצד מה שהיה ממנו החסרון |
|
יהיה הארבעה לשנים על יחס הכפל |
|
והתשעה לששה על יחס המוסיף חצי |
|
והששה עשר לשנים עשר על יחס המוסיף שליש |
|
ועל הדרך הזה |
| The difference is according to the ratio of the natural numbers, starting from two. | ויהיה החלוף על יחס המספרים הטבעיים מתחילים מן השנים |
| Arranging the first of the "others" after the first square, starting from one, then their second after the second square. | אח"כ כאשר תסדר ראשון הזולתיים אחר המרבע הראשון מתחיל מן האחד והשני להם אחר המרבע השני |
| The ratio itself is compound: | הנה יבא זה היחס בעצמו מחבר |
|
|
ויהיה יחס השנים אל האחד כיחס הארבעה אל השנים |
|
ויהיה יחס הששה אל הארבעה כיחס התשעה אל הששה והוא יחס המוסיף חצי |
| וכבר יסודרו תמיד ותהיינה השתי קצוות מבלי יחס מספרי כשיקובץ עם כפל האמצעי מרבע | |
| אח"כ כשתקבץ השתי שורות על סדורם | |
| ותתחיל הנפרדים מן האחד יתילדו מהם המספרים המשלשים על סדורם | |
|
ותמצא כל בעל צלע כאשר יחוסר ממנו צלעו יתילד הזולתי אשר הוא בשכונתו מצד החסרון |
|
וכאשר תוסיף עליו צלעו יתילד הזולתיי אשר הוא בשכונתו מצד התוספת |
| וכאשר תגיע[51] צלע המעקב ממנו ישאר צלעותיו ממנו | |
| וכאשר תקח מושטח בין שני מרבעים תמצא המרבע הראשון יקח ממנו יחס והמרבע השני יחס אחר אמנם ישובו אל היחסים הנמשכים מתחילים מן הכפל אח"כ הדמיון והחצי | |
| ועל הדרך הזה אמרו הנה הנפרדים יתנו סבת ההוא הוא ולקח ה[ההויים] | |
| ולכן יתילדו מהם המרבעים והמעקבים וימצאו במדרגות הנפרדי' מרבע ולא ימצאו במדרגות הזוגות כלל | |
| נשלם המאמר השלישי מן אלארתמיטיקי שבח לאל |
Proportions |
|
| It is a common practice to mention at this point the ratios, their types and their properties. | וכבר פשט המנהג לזכור בזה המקום היחסים ומיניהם וסגלותיהם |
| Some people established many types of relations, reaching up to twenty types and some were satisfied with ten, which were translated ancients. | ומן האנשים מי שיחדש להתיחסויות פנים רבים ויגיעו בהם אל עשרים פנים ומהם שנסתפקו על עשרה והוא המועתק מהקדמון |
| The intention is to be satisfied with mentioning these ten and not tending to bring everything they brought and to mention everything they said, because this has no perfection. | ומכונתי שאסתפק בזכר אלו העשרה ועם ההסתפקות בהם לא תטה נפשי אל הבאת כל מה שהביאוהו וזכרון כל מה שאמרו אותו כי זה ממה שאין שלמות לו |
| It should be known that these are the examined relations, and most of their realization is in the diversity between them and the different properties whose diversity is in one order: | ואתה ראוי שתדע שאלו ההתיחסויות הנבחני' ורוב הגעתם במה שיש ביניהם ההתחלפות והענינים המתחלפים אשר ירוץ התחלפותם על סדר אחד |
|
אם מתדבק כמו יחס א' אל ב' כמו ב' אל ג' |
|
או מתחלק כמו יחס א' אל ב' כמו ג' אל ד' |
| Either their similarity and the uniqueness of their arrangement is in the quantity of themselves or in their quantity according to other. | אם שיהיה התדמותם והתיחדות סדורם בכמות נפשם או כמותם אצל זולתם |
| This is the root and the examined. | וזה הוא השרש והנבחן |
|
והתדמות חלוף המספרים בכמות נפשם הוא כמו שיהיה תוספת זה על זה שוה לתוספת השלישי על הרביעי |
|
כמו תוספת הששה על הארבעה והעשרה על השמנה או הארבעה על השנים |
|
ואלו הם ההתיחסויות המספריים |
|
והדמות חלופי המספרים בכמותם אצל זולתם הרי הוא כמו שיהיה כמות תוספת זה על זה כמו כמות זה השלישי על הרביעי או יהיה כמות זה המתחלף אצל המתחלף אליו אחד |
|
כמו ענין הארבעה אצל השנים בהתחלפות הוא כמו ענין העשרה אצל הששה |
|
וזה הוא ההתיחסות התשברתיי |
| These two are in essence two roots | ושני אלו באמתות שני שרשים |
| When someone examines the property of difference of the relative quantity and the difference of the numerical quantity in the arithmetic proportion [and the geometric proportion], they are found different and no harmony is found at all: | אמנם כאשר יבחן אי ענין חלוף הכמות הצרופיי בחלוף הכמות המספריי בהתיחסות המספריי וענין חלוף הכמות הצרופיי נמצאו מתחלפות והנה לא ימצא הנה הסכמה כלל |
|
המשל בזה שנניח יחס תשברתיי כמו ארבעה ששה תשעה |
|
והנה הכמות המצטרף בהם מתדמה והכמות אשר למספר בעצמו בלתי מתדמה כי החלוף באחד מהם שנים ובאחר שלשה |
|
והנה נניח יחס מספריי כמו ארבעה וששה ושמנה |
|
ותמצא חלוף הכמו' בעצמו שוה וחלוף הכמות בהקש בלתי מתדמה |
|
אבל יהיה ששה לארבעה מוסיף חצי |
|
ושמנה לששה אינו מוסיף כי אם בשליש |
|
וימצאו שני היחסים תמיד נמשכים אבל היחס הגדול מהם בין שני המספרים היותר קטנים והקטן מהם בין שני המספרים היותר גדולים |
|
ויחסם מזה הענין אחד והוא שתבקש מספרים חבורם חבור ישים השני יחסים אשר ביניהם נמשכים וישים הגדול בין הגדולים והקטן בין הקטנים |
|
והנה ימצא יחס אחד על זה התאר כמו יחס מה שבין הששה והארבעה והשלשה ויקרא חבוריי לפי שהתועלת בהשגחת אמצעי זה ההתיחסות אמנם יפול במלאכת החבור והוא המוסיקי כפי מה שתדעהו במקומו |
|
וכבר יעבר שיהיה נקרא חבוריי לפי שיחס הקצוות מחובר מיחס המותרות כפי מה שתדע |
|
ויתחיב לו סגלה שיחס מותר הגדול על האמצעי אל מותר האמצעי על הקטן הוא יחס הקצה הגדול אל הקטן |
|
כמו יחס השנים והוא מותר הששה על הארבעה אל אחד אשר הוא מותר השלשה על השנים[52] |
| From this property that is necessary for this ratio, they observed the examination of the excess of the related terms, and gradually moved from them to other relations and proportions that are useful from the aspect of perfection of the division or multiplication of the ratio. | אח"כ הם התבוננו מזאת הסגולה אשר נתחיבה לזה היחס לבחינת התיחסויות מותר הגבולים המתיחסים והלכו בהדרגה מהם אל התיחסויות ואמצעים אחרים אמנם יועילו מצד השלמות חלוקת היחס או רבויו |
| We will begin with each relation and proportion and say a brief statement about them figuratively: | ונתחיל בהתיחסות התיחסות ואמצעי אמצעי ונאמר בהם דבור קצר על דרך העברה |
|
אם האמצעי התשברתיי הנה אמנם יהיה גדר הכאת הקצוות והנה יהיה גדר מה שיתקבץ משתי הקצוות אחד מהם באחר |
|
וכבר ידעת זה ממקום אחר |
|
וידעת כי כשהיה תמורת האמצעי שני אמצעיים הנה הכאת אחד מהם באחר כהכאת אחת משתי הקצוות באחרת |
| This leads you to the investigation of the mean: | וזה יורה אליך אל דרישת האמצעי |
| It is known in this investigation that the geometric relations are added three by three in the gradual progress of the successive "other" with the successive squares. | וידעת כי בזאת החקירה שאלו ההתיחסויות התשברתיות יתחברו שלשה שלשה בהדרגת הזולתיים הנמשכים עם המרבעים הנמשכי' |
| You know from other places that every two squares may apply these properties. | וכבר ידעת גם כן ממקומות אחרים שכל שני מרבעים אפשר שיפלו הודעת אלו הענינים |
|
אמנם ההתיחסות והאמצעי המספרי באחד או בעשרה והנה שם תמצא אותם מתדבקים באמצעי זה ממה שכבר קדם לך |
|
וידעת ענין המשך היחס וכפילת היחס שלהם והוא שילקח חצי מקבץ השתי קצוות כפי מה שידעת ממרבע האמצעי בכמו מרבע המותר |
| כמו שהכאת השנים בששה הוא השנים בעצמו | |
|
אמנם ההתיחסות והאמצעי החבוריי כבר ידעת התנגדותם למספרי במה שימצא ההתנגדות בהם |
|
והוצאת האמצעי בשנכה החלוף אשר בין הגדול והקטן בקטן ונחלק על מקובצם ונוסיף אותו על הקטן ויצא האמצעי |
|
כמו שיהיה תשעה ותחלק על מקובץ השלשה והששה והנה יצא אחד ותוסיף אותו על השלשה ויהיו ארבעה |
| וכאשר יהיה אצלך האמצעי והגדול ותרצה שתמצא הקטן תעיין אל מותר מה שביניהם כמה הוא מן האמצעי כשנחלק עליו האמצעי פעם אחרת והנה מה שיצא נגרע אותו מן האמצעי | |
| וכאשר יהיו הקטן והאמצעי ידועים אצלך ותרצה הגדול תחלק האמצעי על המותר ותגרע ממנו אח"כ תחלק עליו ומה שיצא תוסיף אותו על האמצעי | |
|
ומסגלות זה ההתיחסות שהכאת מקובץ השני קצוות באמצעי כמו כפל אחת הפאות באחרת |
| וג"כ הנה הכאת האמצעי בגדול כמו כפל האמצעי בקטן בשעור הכפלת אחת משתי הקצוות אל האחרת | |
| וכבר חשבו אנשים שזה היחס אמנם נקרא חבוריי לפי שמותרותיו שחלוקותיו אינם בגבולים לבדם ולא במותרות לבדם אבל קצת בזה וקצת בזה וכאלו נפל בזה חבור וזה טורח | |
| וכבר אמרו מה שהוא יותר חזק הטרח מזה | |
| אמנם ההתיחסויות אשר אחר אלו הנה מהם שלשה נודעו ראשונה ומהם ארבעה נודעו שנית ומהם התיחסויות אין מכונתנו שנשגיח אליהם | |
| ואלו הארבעה יודעו בשלישי והרביעי והחמישי והששי | |
| ויקרא הרביעי המתנגד לפי שהוא מנגד לחבוריי באשר הוא הושם בצד | |
| The ratio of the excess of the mean over the smaller to the excess of the greater over the mean is as the ratio of the greater to the smaller
|
והנה יחס מותר האמצעי על הקטן ומותר הגדול על האמצעי כיחס הגדול אל הקטן |
|
כמו חמשה עשר ושמנה וששה |
| והוצאתם בהכאת המותר שבין שתי הקצוות הקטנו' בקטן והחלוקה על מקובצם והכפלת מה שיצא מן הגדול הנה הוא האמצעי סגלותיו שהכאת הגדול באמצעי כפל הכאת באמצעי התיחסות | |
|
והאמצעי החמישי שיהיה האמצעי אצל הקטן כמו מותר השני קטנים אצל מותר השני גדולים |
| ומספרם כאלו נגזר בזה התשברתיי | |
| ודרישת זה האמצעי שתוסיף הקטן על הגדול ותחלק מה שיתקבץ חלוקה תהיה הכאת אחד מהם באחר כהכאת הנשאר מהגדול אחר השלכת הקטן ממנו בקטן וזה נקל למי שידע היחס | |
| ואם אפשר זה זה ואם לא הנה השאלה בטלה | |
| ומה שיצא יחוסר הקטן מהגדול ממנו ומה שנשאר הנה הוא האמצעי | |
| ומסגלותיו שהכאת הגדול באמצעי כפל הכאת הגדול בקטן | |
| ומזה שהאמצעי מהם בהתיחסות הכפליי נגדר לעולם וגדרו הקטן | |
| והקצה הגדול קטן ממקבץ הנשארים באחד | |
|
הששי שיהיה הגדול אצל האמצעי כמו מותר השני קטנים |
| ודמיון זה | |
| והוצאת האמצעי כשנחסר הקטן מן הגדול כמה שיצטרך שיתוסף על הגדול והתוספת עד שיהיה הכאת זה בכל המקובץ מן השרש והשני תוספת כמו המושטח אשר שמר והנה מקובץ השני תוספות הוא האמצעי | |
| ואם נשבר הנה השאלה בטלה | |
| וג"כ הנה אתה כאשר תגרע ותכפול גדרו ותגרע ממנו המוכה ראשונה בעצמו ומה שנשאר תוסיף אותו על הקטן | |
| וכבר יחויב לו מן הסגלות שההתיחסות כשהיה על יחס הדמיון והחלק יהיה האמצעי נגדר | |
| וכאשר יצטרף אליו גדרו יהיה מקבצו הקצה הגדול והקצה הקטן קטן ממנו | |
|
אמנם הארבעה אשר ידעת באחרונה הנה הראשון שבהם הוא השביעית שיהיה יחס המותר בין השתי קצוות אל המותר בין שני הקטנים כיחס הגדול אצל הקטן |
|
המשל בזה הששה והשמנה והתשעה |
|
|
והוצאת האמצעי שלו בהכאת הקטן במותר אשר בינו ובין הגדול וחלוקת המתקבץ על הגדול ותוספת היוצא על הקטן ומה שהגיע הנה הוא האמצעי |
|
והשמיני שיהיה יחס הגדול אל הקטן כיחס מותר שתי הקצוות אל מותר השני גדולים |
|
המשל בזה ששה שבעה תשעה |
| והוא הפך השביעית | |
| והוצאת האמצעי שלו הפך הוצאת זה האמצעי | |
|
|
וזה בהכאת הקטן במותר אשר בין שתי הקצוות וחלוקת היוצא על הגדול ומה שיצא נגרע אותו מהגדול ומה שישאר הנה הוא האמצעי |
|
התשיעי שיהיה יחס מותר הקצוות אל מותר השני קטנים יחס האמצעי אל הקטן |
| כמו הוצאת האמצעי שלו כשנגרע הקטן מהגדול ונחלק השני חלוקה תהיה אחד החלקים אל האחר כיחס האחר אל הקטן אם אפשר ונפיל החלק הראשון מהגדול ומה שישאר הנה הוא האמצעי | |
|
|
ובידך שתקבץ הכאת המותר בקטן אל מרבע חצי הקטן ותקח גדרו ותוסיפהו על חצי הקטן |
| וזה ההתיחסות כאשר יהיה על יחס הדמיון והחלק יהיה הקצה הקטן מרבע תמיד | |
| והתיחסות והאמצעי העשירי שיהיה יחס מותר השתי קצוות אל מותר השני גדולים | |
|
|
כמו יחס האמצעי שלו שתקח מותר מה שבין הקצוות מוכה בקטן מחוסר ממרבע חצי הגדול ותקח גדר זה תוסיפהו על חצי הגדול |
| אלו צריכים בשתי קצוות עם התשברתיי תמיד ולא עם השביעי והשמיני ולא עם החבוריית אל שיהיה הגדול הפך הקטן כמו הרביעי אלא שיהיה הגדול גם כן הפך הקטן והתשבריית לא השמיני ימצאו עם החבוריים ולא עם הרביעי ולא עם השביעי ולא עם השמיני ולא עם התשיעי | |
|
וכאשר הונחו לנו השמנים והעשרים שני גבולים |
|
יהיו החמשים ביניהם אמצעי מספריי |
|
וארבעים אמצעי תשברתיי |
|
ושנים ושלשים אמצעי חבוריי |
|
ושמנה וששים אמצעי רביעי |
|
וחמשה ושלשים אמצעי שביעי |
|
וחמשה וששים אמצעי שמיני |
| The fifth, the sixth, the ninth, and the tenth are extracted: | וכבר הוצאו החמישי והששי והתשיעי והעשירי |
|
והנה נניח ראשון גבולי ההתיחסות החמישי והם |
|
וכאשר חוסר מהקטן אחד ונוסף על הגדול היו והוא ההתיחסות הששי |
|
וכאשר נוסף על כל גבול שנים עד שיהיו יצא ההתיחסות התשיעי |
| וכאשר מן האמצעי אחר זה מה שאמרנוהו בחכמת הארתמיטיקי | |
| וכבר הנחנו ענינים היה המנהג לחברם בזה המקום יוצאים מסדר המלאכה | |
| וכבר אצל המעשה כמו החבור וההקבלה והקבוץ והחלוק ההוריי ומה שירוץ מרוצתם | |
| והראוי בכמו אלו שיזכרו בענפים והנה נסתפק הנה על ההגעה הנזכרת ונקח בחכמת המוסיקי בע"ה | |
| What is done with the number in relation and comparison: | הנעשה במספר על זה ההצטרפות וההקש |
|
כשהוקש אחד משני מספרים אל האחר הנה לא ימלט מאשר יהיו שוים או מתחלפים |
|
ואם היו שוים היה היחס ביניהם יחס השווי והיושר |
|
וידמה שזה היחס הוא התחלה טבעית לשאר היחסים ושהוא אשר אליו יותך כל יחס |
|
ואיך יהיה זה בסדור הניחו בכלם נזכרהו עוד אחר זה |
|
ואם היו מתחלפים לא ימלט אם שיהיה יחס היותר רב שבהם אל היותר מעט יחס הדמיונים כעשרים והשלשים אצל העשרה |
|
או יחס הדמיון המוסיף חלק כעשרה אצל השמנה |
|
או יחס הדמיון המוסיף חלקים כעשרה אצל הששה |
|
או יחס הדמיונים המוסיף חלק כעשרה אצל השלשה |
|
או יחס הדמיוני המוסיף חלקים כחמשה עשר אצל הארבעה |
| These are the categories of the numerical ratios when the greater is related to the smaller. | ואלו הם חלוקות היחסים המספרים כאשר הוקש בהם היותר רב אל היותר מעט |
| When you know this, you know the property of the smaller to the greater. | וכאשר ידעת זה ידעת ענין המעט אצל הרב |
| The less is called the opposite. | ויקרא היותר מעט המקביל |
| The less in the sesquialter ratio, for example, is called the opposite to the sesquialter ratio. | ויקרא היותר מעט ביחס הדמיון המוסיף חצי דרך משל המקביל לדמיון המוסיף חצי |
| In the sesquitertian ratio - the opposite to the sesquitertian ratio. | וביחס הדמיון המוסיף שליש המקביל לדמיון המוסיף שליש |
| And so on for the others. | וכן בשאר |
| It is also [said?] about the less, as beneath so and so, it is said sub-super so and so sub-multiple so and so. | וכבר יולץ ג"כ מן היותר מעט כאשר תחת פלוני ויאמר לו אשר תחת הדמיון המוסיף ככה או הדמיונים המוסיפים ככה |
| The arrangement of the production of all of these ratios is as mentioned. | והסדור בהמצאת אלו היחסים כלם כפי מה שנזכרהו |
|
אם הדמיונים הנה הענין בהם מבואר |
|
והראשון שבהם השניי אחר ימשך אליו השלישיי אחריו הרביעיי אחריו החמישיי וכן תמיד |
|
וזה היחס מתחיל מהשנים והולך על המשך המספר עד אין תכלית |
| The other ratios are found by taking the number by which the additional part or parts are denominated. | אולם היחס אשר זולתו זה הנה אתה תמצאהו בשתקח המספר אשר בשמו יקרא החלק המוסיף או החלקים |
|
ואם תרצה הדמיון המוסיף חלק תוסיף זה החלק על זה המספר אשר יקרא בשמו ויתקבץ לך מה שתרצה |
|
דמיון זה כשתרצה הדמיון ושמינית |
|
הנה תקח המספר אשר בשמו יקרא זה החלק והוא שמנה ותוסיף עליו שמיניתו וזה אחד ויתקבץ מהם תשעה |
|
ויהיה יחס תשעה אל שמנה יחס הדמיון המוסיף שמינית |
|
וכן ג"כ אם תרצה יחס הדמיון המוסיף חלקים |
|
כמו המוסיף שתי חמישיות |
|
הנה תקח חמשה כי בשמו נקראים ותוסיף שני חמישיותיו והוא שנים ויתקבץ שבעה |
|
ויחס שבעה לחמשה יחס הדמיון המוסיף שתי חמישיות |
|
ואולם הדמיונים המוסיפים חלק או חלקים הנה תמצאהו כשתכפול המספר אשר בשמו יקראו החלק או החלקים כמספר הדמיונים המונחים ותוסיף על מה שיתקבץ החלק המבוקש מזה המספר או החלקים ויהיה הכלל כמבוקשך |
|
דמיון זה אם תרצה השלשה דמיונים המוסיף חומש |
|
הנה תקח חמשה ותכפלהו במספר הדמיונים שהם שלשה ויעלה חמשה עשר ותוסיף על זה חומש החמשה שהוא אחד ויעלה ששה עשר והוא היותר רב |
|
ויחסו אל החמשה שהוא היותר מעט יחס השלשה דמיונים המוסיף חומש |
|
וכן תעשה ביחס הדמיונים המוסיף חלקים |
|
|
שתוסיף כאן שנים על החמשה עשר ויהיו שבעה עשר |
|
ויחסם אל חמשה השלשה דמיונים המוסיף שתי חמישיות |
| When the parts are reduced to one part | וכאשר ישובו החלקים אל חלק אחד |
|
כמו שישובו השתי ששיות אל השליש |
|
|
והארבעה שמיניות אל החצי |
|
ומה שדומה לזה |
| Their rule is according to the rule of what they are reduced to. | הנה משפטם בסדור משפט מה שישובו אליו |
| Also, if the superparticular or the superpartient involves fraction of fraction: | וג"כ אם יהיו המוסיף על הדמיון או הדמיונים חלק חלק |
|
כמו שתות השתות |
|
|
וחומש החומש |
|
|
או כשליש העשור |
|
|
וחומש השביע |
|
ומה שהוא יותר דק מזה |
|
|
או אם היה חומש ושתות |
|
|
או שליש ורביע |
|
ומה שדומה לזה |
| All of them are reduced to one part or parts. | הנה אלה כלם ישובו אל החלק האחד או החלקים |
| The fraction of fraction is also a fraction: | וזה כי חלק החלק הוא ג"כ חלק |
|
|
כמו שתות השתות שהוא חלק מששה ושלשים |
|
|
וחומש החומש שהוא חלק מחמשה ועשרים |
|
|
וכן שליש העשור שהוא חלק משלשים |
|
|
וחומש השביע שהוא חלק מחמשה ושלשים |
|
|
וכן השליש והרביע שבעה חלקים משנים עשר |
|
|
והחומש והשתות אחד עשר חלקים משלשים |
| The arrangement discussed concerning the reduction of these ratios to equality: | ואולם הסדור אשר דברנו[53] בהשבת אלו היחסים אל יחס השווי |
|
הוא שנניח זה היחס בשלשה גבולים יהיה יחס הגדול מהם אל האמצעי הוא בעינו יחס האמצעי אל הקטן |
|
ונחסר לעולם מן הגדול כפל האמצעי מחוסר ממנו הקטן |
|
ונחסר מהאמצעי כמו הקטן |
|
ונניח הקטן כמו שהוא |
|
ואם יהיו שוים הגבולים השלשה הנה המבוקש ואם לא תשוב ותעשה כמו מה שעשינו ראשונה עד שיהיו שוים |
|
ודמיון זה ביחס הארבעה דמיוני שיהיה הגדול ס"ד והאמצעי י"ו והקטן ד' |
|
|
הנה כשכפלנו האמצעי ונחסר ממנו הקטן נשארו כ"ח וכאשר נחסר זה מן הגדול נשארו ל"ו |
|
|
וכשנחסר הקטן מן האמצעי נשארו י"ב |
|
|
ונשאיר הקטן בענינו |
|
ויהיו אלו המספרים השלשה אשר הם ל"ו י"ב ד' על יחס השלשה דמיונים |
|
|
וכשנשוב ונחסר ג"כ מן הל"ו כפל הי"ב מחוסר ממנו הד' וזה עשרים נשארו י"ו |
|
|
אחר כן נחסר מן הי"ב הד' נשארו ח' |
|
|
ונשאיר הד' כמו שהם |
|
ויהיו המספרים השלשה אחר זה י"ו ח' ד' על יחס הכפל |
|
|
וכשנשוב שלישית ונחסר כפל הח' מחוסר ממנו הד' וזה י"ב מן הי"ו |
|
|
אח"כ נחסר הד' מן הח' |
|
|
יהיו שוים הגבולים הנשארים ויהיה כל אחד מהם ארבעה |
|
ואתה רואה איך שב המרבע אל המשלש אשר הוא יותר פשוט ממנו |
|
אח"כ המשלש אל השניי אשר הוא יותר פשוט ממנו |
|
ואחר הותך אל יחס השווי |
|
עוד נמשיל ביחס הדמיון וחלק ויהיה החצי |
|
ונשים השלשה מספרים אשר הם על זה היחס י"ח י"ב ח' |
|
|
כפלנו הי"ב וחסרנו ממנו ח' נשארו י"ו וחסרנו זה מי"ח ונשארו ב' |
|
|
וכשנחסר ח' מן י"ב נשארו ד' |
|
|
ונניח ח' כמו שהוא |
|
ויהיה השלשה גבולים ב'ד'ח' על יחס הכפל |
|
|
ונשוב ונחסר מהח' שהוא הגדול כפל האמצעי שהוא ד' מחוסר ממנו הקטן שהוא ב' וזה ו' נשארו מהגדול ב' |
|
|
וכשנחסר מהאמצעי ב' נשאר גם כן ב' |
|
|
ונשאיר הקטן ב' כמו שהוא וזה יחס השווי |
|
ואתה רואה ג"כ איך שב יחס הדמיון והחלק אל יחס השווי |
|
ואיך יותך ראשונה אל יחס הכפל השניי ואחר יותך זה יחס אל יחס השווי |
| The types of proportions: | ומיני המתיחסים |
|
אם שיהיו על צד הדבקות והם אשר יהיה האמצעי משותף בין שתי הקצוות ילקח בהתיחסות פעם נמשך ופעם קודם |
|
וגבוליו תמיד שלשה לא פחות ולא יתר |
|
כאמרנו יחס א' אל ב' כיחס ב' אל ד' |
|
ואם שיהיה על צד ההבדל ולא יהיה בזה אמצעי אחד משותף אבל אמצעיים |
|
כאמרנו יחס א' אל ב' כיחס ד' אל ח' |
|
והגבולים בזה היחס תמיד יותר משלשה |
| The types of proportions are numerous, of them Nicomachus has mentioned only ten types in his book. | ומיני המתיחסים רבים זכר מהם ניקומכוש בספרו עשרה מינים לבד |
| A sect of the ancients endeavored in the investigation of the additional to them and found ten others, so there were twenty types. | ויחתרו כת מן הקדמונים בדרישת מה שנוסף עליהם ומצאו עשרה אחרים והיו בהם מיני היחס עשרים מינים |
| Yet, the most beneficial among them in the sciences are mostly only three, which are the arithmetic, the geometric, and the harmonic proportions. | והמועילים בהם בחכמות על הרוב שלשה לבד והם המתיחסים המספריי' והתשברתיים והחבוריים |
| First we will deal with the mentioning of these, then the mentioning of the others among the first ten types will follow. | ונתעסק בזכרון אלו ראשונה אח"כ ימשך להם זכרון הנשארים מן העשרה מינים הראשונים |
| As for the ten additional, to which the ancients strived, we have no business in mentioning them because they are hardly found. | והעשרה הנוספים אשר טרחו בהם הקדמונים אין לנו עסק בזכרונם הנה למעוט מציאותם |
|
ואולם המתיחסים המספריים הם המתיחסים הנופלים בין גבולים אם שלשה או יותר מזה נמשכים על שווי מה שביניהם מהתוספת והיתרון |
|
כשנים והשלשה והארבעה המוסיפים אחד אחד |
|
וכחמשה והשבעה והתשעה המוסיפים שנים שנים |
|
ואין היחס בין אלו המספרים אחד בכמות רצוני היתרון |
|
כי יחס הארבעה אל השלשה יחס הדמיון המוסיף שליש |
|
ויחס השלשה אל השנים יחס הדמיון המוסיף חצי |
|
ויחס התשעה אל השבעה יחס דמיון המוסיף שתי שביעיות |
|
ויחס השבעה אל החמשה יחס הדמיון המוסיף שתי חמישיות |
|
וכן יהיה ג"כ בנבדלים |
|
כמו השנים והחמשה והארבעה והשבעה והשמנה והאחד עשר |
|
כי היחס אשר בין אלו המספרים הששה יחס מספריי על צד ההבדל והגבולים בו מוסיפים בכמות אחת והוא השלשה |
|
ואולם ההתיחסות אינו אחד |
|
לפי שיחס האחד עשר אל השמנה יחס הדמיון המוסיף רביעית ושמינית |
|
ויחס השבעה אל הארבעה יחס הדמיון המוסיף חצי ורביעית |
|
ויחס החמשה אל השנים יחס הכפל המוסיף חצי |
|
ומסגלות אלו המתיחסים וזה האמצעיות שמקובץ הקצוות במתדבקים מהם כפל האמצעי ובנבדלים שוה למקובץ האמצעיים |
|
ומסגלותם גם כן שהיחס שבין שני הגבולים הקטנים במדובקים מהם והנבדלים יותר גדול תמיד מהיחס אשר בין שני גבולים הגדולים |
|
ומהם שהכאת אחת הקצוות באחרת יותר מעט תמיד ממרובע האמצעי במדובקים כמו מרבע התוספת |
|
ומאלו הסגלות וממה שקדם להם מהידיעה בסגלות המספרים יודע האמצעי באלו המתיחסים מצד שתי הקצוות |
|
וזה במתדבקים כאשר ילקח חצי מקבצם ומה שהיה הוא האמצעי |
|
לפי שכל מספר שוה לחצי מקובץ שתי פאותיו כמו שידעת |
|
ואמנם בנבדלים הנה לו יוגבלו שני האמצעיים מהידיעה בשתי הקצוות לבד אבל כבר אפשר שימצאו ביניהם אמצעיים רבים |
|
ואם יהיו האמצעיי' נעלמים יחד והיה מקובצם ידוע או מותר מה שביניהם ידוע ויחס אחד מהם אל האחר ידוע הנה אפשר מציאות כל אחד מהם על מנינו |
|
ואם לא יהיה דבר מאלו הדברים ידוע יהיה דרך הוצאתם ומציאות כל אחד מהם ממה שאין דרך לזכרו הנה |
|
ואולם המתיחסי' התשברתיים הם אשר יהיה היחס אשר בין גבוליהם אחד והמותרות מתחלפות בחלוף המספריים |
|
כמו האחד והשנים והארבעה במתדבקי' |
|
והאחד והשנים והשלשה והששה בנבדלים |
|
|
וזה שיחס ד' ב' כיחס ב' א' |
|
|
והמותר בין ד' ב' יותר ממותר שבין ב' א' |
|
וכן בו' ג' וב' א' שיחסם אחד ומותרם מתחלפים |
|
ומסגלות אלו המתיחסים וזה האמצעיות שהכאת אחת הקצוות תמיד באחרת שוה למרבע האמצעי במתדבקי' ולהכאת אחד האמצעיים באחר בנבדלים |
|
ולזה היה מציאות האמצעי והוצאתו אם במתדבקים הוא כאשר ילקח שרש הכאת אחת הקצוות באחרת ומה שהיה הוא האמצעי |
|
ואם בנבדלים הנה יצטרך עם הידיעה בכל אחת מהקצוות שיהיה אחד משני האמצעיים ידוע כדי שנדע מזה הצד האמצעי האחר וזה בחלוק הכאת אחת הקצוות באחרת עליו |
|
ואם היו מוסכלים יחד יהיה מקובצם ידוע או מותר מה שביניהם ידוע או יחס אחד מהם אל האחר ידוע הנה אפשר מציאות כל אחד משניהם על מנינו |
|
ואם לא יהיה אחד מאלו הדברים ידוע יהיה דרך הוצאותם ומציאות כל אחד מהם ממה שאין דרכו שיחובר הנה |
|
ואולם ההתיחסות החבוריי הוא אשר יהיה בו יחס גדול שבגבוליו אל הקטן כיחס מותר מה שבין הגדול והאמצעי אל מותר מה שבין האמצעי והקטן |
|
כמו השלשה והארבעה והששה |
|
|
כי יחס הששה אל השלשה כיחס מותר מה שבין הששה והארבעה והוא שנים אל מותר מה שבין הארבעה והשלשה והוא אחד |
|
והצורך אל זה המין ממיני המתיחסים נוגע בחכמת חבור הלחנים הנקרא המוסיקי לפי שיחס המרחקים המסכימים בו אמנם ימצאו בזה המין לבד כפי מה שתאמר עליו אחרי זה ולכן נקרא חבוריי |
|
והוצאת הגבול האמצעי מגבולי ההתיחסות הזה הוא כשנכה לעולם מותר מה שבין הקצוות בקטן שבגבולים ונחלק מה שהתקבץ על מקובץ שתי הקצוות ונוסיף מה שיצא על גבול הקטן ומה שהתקבץ הנה הוא הגבול האמצעי |
|
דמיון זה אם נדע השלשה והששה |
|
|
הנה המותר שלשה נכה שלשה בשלשה יהיה תשעה ומקובץ הקצוות ג"כ תשעה וכשנחלק תשעה על תשעה יעלה אחד לבד נוסיף האחד על השלשה שהוא גבול הקטן ויהיה ארבעה והוא האמצעי |
| These three types of proportions are used in the sciences, and the received is of benefit in them. | הנה אלו השלשה ממיני המתיחסים הם הנעשי' בחכמות והמקובל תועלת בהם |
|
ואולם המין הרביעי הוא אשר יהיה בו יחס הגדול אל הקטן כיחס מותר מה שבין שני הגבולים הקטנים אל מותר מה שבין שני הגבולים הגדולים |
|
כמו השלשה והחמשה והששה |
|
וזה המין מקביל למין החבוריי |
|
ויסגלהו שמוכה הגדול באמצעי כפל מוכה האמצעי בקטן |
|
כמו שיהיה בכאן מוכה ששה בחמשה והוא שלשים כפל מוכה שלשה בחמשה והוא חמשה עשר |
|
ואולם המין החמישי הוא אשר יהיה בו יחס האמצעי אל הקטן כיחס מותר מה שבין שניהם אל מותר מה שבין האמצעי והגדול |
|
כמו השנים והארבעה והחמשה |
|
ויסגלהו שמוכה הגדול באמצעי כפל הכאתו בקטן |
|
כמו שהכאת החמשה בארבעה והוא עשרים כפל הכאת חמשה בשנים אשר הוא עשרה |
|
ואולם המין הששי הנה הוא אשר יהיה בו יחס הגדול אל האמצעי כיחס מותר מה שבין האמצעי והקטן אל מותר מה שבין האמצעי והגדול |
|
כמו האחד והארבעה והששה |
|
ואולם המין השביעי הנה הוא אשר יהיה בו יחס הגדול אל הקטן כיחס מותר מה שבין שניהם אל מותר מה שבין האמצעי והקטן |
|
כמו הששה והשמנה והתשעה |
|
ואולם המין השמיני הנה הוא אשר יהיה יחס הגדול אל הקטן כיחס מותר מה שבין הקצוות אל מותר מה שבין הגדול והאמצעי |
|
כמו הששה והשבעה והתשעה |
|
ואולם המין התשיעי הנה הוא אשר יהיה בו יחס האמצעי אל הקטן כיחס מותר מה שבין הקצוות אל מותר מה שבין האמצעי והקטן |
|
כמו הארבעה והששה והשבעה |
|
ואולם המין העשירי הנה הוא אשר יהיה בו יחס האמצעי אל הקטן כיחס מותר מה שבין שני הקצוות אל מותר מה שבין האמצעי והגדול |
|
כמו השלשה והחמשה והשמנה |
| הנה אלו הם המינים העשרה ממיני המתיחסים שיספיק לנו זכרונם כי אין לאחד מהם מבא בחכמה זולתי מה שזכרנום לפנים ואולם הביאונום על צד שלמות החלוקה והמשכתה וספירת מה שיגיעו אליו צדי ההתיחסות נשלם זה והתהלה לאל ית' שמו |
Music |
|
| האופן הרביעי מן החלק השני בחכמת המוסיקי | |
| אמר אמיה בן עבד היקר אבו אלצלת הנה נסתם מה שקדם מאופני הלמודים באומר בחכמת המוסיקי וזה ספר כבר ספרנוהו לו כמו שעשינו בשאר אופנים וחלקנוהו חלקים שמנוהו הראשון מהם בהתחלות זה האופן ומה שירוץ מרוצת ההתחלות ויכנס בה מן ההכנות וההצעות והמשכנוהו במאמר בספירת הנעימות והמרחקים והסוגים והקבוצים מרובם והמזגתם ואיכות סדר הסוגים בקבוצים עוד בהמצאתם מוחשים בכלים המלאכותיים ובחלקי הכלים ובמיניהם עוד באומר בהעתקות וחלקי הנפילות ומיניהם והלכנו במה שהבאנוהו מזה צד הדרך אשר הלכנו בזה הספר מהבאת מה שאין די זולתו וחסר מה שאין צריך לו ובאל נעזר | |
| המאמר הראשון מזה האופן שני פרקי פרק ראשון בהתחלות זה האופן ומה שירוץ מרוצת ההתחלות ויכנס בה מן ההכנות למלאכת המוסיקא מלאכה נושאה הנעימות וכונתה העיון בם מצד מה שיתקרבו ויתרחקו ובאיכות חבורם כדי שיהיה מהם לחן ותתחלק אל עיונית ומעשית והעיונית היא אשר מדרכה שתחקר מהנעימות ומה המסכים מהם ובלתי מסכים ותעיין בחבור המסכים מהם עד יתכן שיהיה המחובר מהם לחן ותחקר מאי זה מהם יהיה יותר טוב ויותר שלם ומן הדברים אשר יהיו בם יותר טובות ויותר שלמות ותתן עלות זה כלו וסבותיו יתנו סבת ההוא הוא ולכן יתילדו מהם המרבעים והמעקבים וימצאו במדרגות הנפרדים מרבע ולא ימצאו במדרגות הזוגות כלל נשלם המאמר השלישי מן הארתמיטיקי ושבח לאל יתעלה שמו וכבר פשט המנהג לזכור בזה המקום היחסים ומיניהם וסגולותיהם ומן האנשים מי שיחדש להתיחסות פנים רבים ויגיעו בהם אל ב' פנים ומהם שנסתפקו על עשרה והוא המעתק מהקדמון ומכונתי שאסתפק בזכר אלו הי' ועם ההסתפקות בהם ונשלם הספר הזה ספר הארתימיטיקא לאמיה בן עבדו אבו אלצלת היקר תדרוש בסגולות המספרים קצר והוסיף על ספר ניקומאכוש הגרישיני הפיתגוריי לידי הצעיר באלפי הדל אליאו גבה בבר אליעזר יצ"ו בשנת
| |
Notes
- ↑ MS St. Petersburg beginning
- ↑ marg. א"ב אם רצה בזה שלא יביא אל נגדר בהקוות השני או שלא יביא אל נגדר באי זה הקוות שיהיה הוא נכון ואולם אם רצה בזה שלא יביא אל נגדר לעולם אין זה צודק כי השלשה אחר י"ג הקוויות יביא אל נגדר אצל פ"א וגם זה יצדק בחמשה ושאר המספרים כאשר תוציא קום
- ↑ marg.א"ב למעלה קרא נגדר ראשון האחד ובכאן קרא נגדר ראשון התשעה והוא הנכון כי האחד אינו מספר ודבר למעלה דרך העברה והסדר הוא שהראשון שבנגדרים הנפרדים תשעה והשני כ"ה והשלישי מ"ט ועל הדרך הזה
- ↑ marg. בל"ד מט'
- ↑ והנה
- ↑ marg. כי לפי זה יחויב שיהיו השנים נמנים במדרגות זוג הזוג
- ↑ marg. א"ב לפי זה יהיו השנים זוג הנפרד וזה כי הארבעה זוג הזוג וכאשר נגרע מהם הזוג הראשון שהוא שנים ישארו שנים ואם שנים זוג הנפרד המאמר צודק ולא בזולת זה ובזה תמה כי במקומות מהספר נראה שהוא לוקח השנים במספר זוג הזוג ובמקומות במספר זוג הנפרד
- ↑ marg. פי' כמו שמנה שהוא זוג הזוג וחלקיו שהם חצי ורביע ושמינית והם ד'ב'א' והגעתם שבעה
- ↑ marg. ירצה לפי שיש ג' מיני יחסי' יחס מדותיי והוא שהשעורי' מתיחסי' והמותרי' בלתי שוי' ויחס מספרי והוא שהשעורי' בלתי מתיחסי' והמותרי' שוים יחס מוסיף והוא שהשעורי' בלתי מתיחסי' והמותרי' בלתי שוי' אבל יחס השעור הא' אל הג' כיחס מותר מה שבין השעור הא' אל הב' אל מותר מה שבין השעור הב' אל השעו' השלישי כמו מספרי' ג'ד'ו' א"ב ר"ל
- ↑ marg. ירצה כשנקח מהם חלקי' מתיחסי' על יחס א' הן לתוספת הן למגרעת כמו עד"מ שנקח החצי או הרביע מכל א' מהם או כפל או ג' כפלי כל א' מהם ודומה לזה וישמרו תמיד יחס הכפל עצמו.. א"ב ר"ל
- ↑ marg. מל"ה מט'
- ↑ marg. א"ב כדי זה המשפט להביא בו משל והנה נקבץ זוגי הזוג מספרי ב' ד' והאחד עמהם ויהיו ז' והוא מספר ראשון ונוסיף עליו אחרון המקובצי' והוא ד' ויהיו י"א וגם הוא ראשון ונחסר ממנו ר"ל מז' המספר אשר לפני אחרון המקובצים והוא ב' וישאר ה' וגם הוא ראשון ונכה י"א והוא הנוסף בה' שהוא המחוסר ויעלה נ"ה הנה הכאת נ"ה באחרון המקובצים והוא ד' יביא ר"ך והוא המספר אשר לו אוהב והוצאת אוהבו שנוסיף הכאת מקובץ הנוסף והחסר הנזכרי' והוא י"ו בד' שהוא אחרון המקובצים שיעלה ס"ד על ר"ך שהוא האוהב הראשון ויהיה רפ"ד שהוא האוהב השני ושניהם נאהבים ועל הדרך הזה
- ↑ marg. ר"ל שיחלק יותר מפעם אחת לא זוג הזוג שנאמר ביחוד על המתחלק תמיד עד האחד
- ↑ marg. לא הבנתי זאת הסגולה ואולי ירצה שקבוץ כל מספרי זוג הנפרד על הסדר שוה לכפלי המרובעי' הטבעיים על הסדר כי קבוץ מספרי ב' ו' שוה לכפל מרובע ב' וקבוץ ב' ו' י' שוה לכפל מרובע ג' וקבוץ ב' ו' י' י"ד שוה לכפל מרובע ד' וכן תמיד על זה הסדר א"ב ד"ל
- ↑ marg. ר"ל ג' ימנה ט' בג' וימנה ט"ו בה' וימנה כ"א בז'
- ↑ marg. הה' ימנה ט"ו בג' וכ"ה בה' ול"ה בז'
- ↑ marg. א"ב ר"ל כל מרבע מספר ראשון בהקש אל מרבע מספר ראשון
- ↑ marg. מסוף ט' לאקלידס
- ↑ marg. א"ב ר"ל כי יציאת ששה היתה כאשר לקחנו שנים זוג הזוג והאחד ונתקבץ שלושה מספר ראשון והכינו אותו בשנים ויצא ששה מסוף ט' לאקליד'
- ↑ marg.א"ב ר"ל שהבית הראשון מהשורה השלישית על הראשון מהשנית יוסיף א' והשני על השני ב' על סדר המספרי' הנמשכים
- ↑ marg. ר"ל בשורה הראשונה תמצא שכל שלישי לאי זה ראשון שיהיה יוסיף בשנים כמו ג' לא' וד' לב' וה' לג' ובשורה השנית יוסיף בארבעה ובשלישי' בששה
- ↑ marg. א"ב צ"ע בזה שורה אחת כי יש טעות בלשון ובסברא אפשר לכוין הלשון על צדדים רבים ולכן לא תקננו כי לא יודע אל איזה כיון המחבר
- ↑ marg.לפי שמקובץ כפל השטחים מרבע בתוספת אחד
- ↑ marg.כמו כפל ד"ט שהוא כ"ו וכ"ה מרבע
- ↑ marg.ר"ל מבלי בחינה בין הגמור והבלתי גמור
- ↑ marg. א"ב לפי שמדרגתם ג"ה ד"ו ה"ז וג"ה יתוסף תמיד בג"ה וד"ו בד"ו וה"ז בה"ז הנה תוספת החסרים והנוספי' אינו רק באחד אחד
- ↑ marg. א"ב צ"ע כי כפי המשך המשלים נראה שהנכון שיאמר הדמיונים והחלק או הכפל והחלק
- ↑ marg. ר"ל ביחס הכפל וחצי
- ↑ marg. א"ב נראה שהרצון הוא כפל מה שהיה בדמיון ושליש כי לא יצדק זה בכפל ושליש במוסיפי' כי אם בחסרים
- ↑ marg. א"ב ר"ל שיחס כ"א אל י"ט וי"ט לי"ז וי"ז לט"ו הם יחסי הדמיון ושני חלקים והם גמורים וכן השאר
- ↑ marg.א"ב ר"ל שיחסי י"א אל י' וי' אל ט' וט' אל ח' הם יחסי הדמיון המוסיף חלק וכן השאר
- ↑ marg. א"ב ר"ל הלוח הגדול הנרשם למעלה בבתי עשר על עשר
- ↑ marg. ר"ל אם נקח הא' המדרגות החצי
- ↑ marg. ר"ל האמצעית
- ↑ marg. א"ב ר"ל בכח כי מספר החמשה עשר שהוא מספר המשלש החמישי אינו משלש בפעל עד שיסודרו סדור ידמה לתמונה המשלשת וישים התושבת ה' ועליהם ד' ועל הד' ג' ועל הג' ב' ועל הב' א'
- ↑ marg. א"ב ר"ל לפי שהאחד אינו מספר עם היותו מרבע כאשר נזכר למעלה אבל לפי שהאחד הוא הנפרד הראשון יצדק כמו כן אמרנו שמרבע האחד יתילד מדבקותו אל עצמו ואם לא יפול בזה מלת קבוץ
- ↑ יחזקאל א, יד
- ↑ marg. א"ב מופת זה המשפט מד' ממאמר שני לאקלידס
- ↑ marg. א"ב כמו כפל ב' וא' עם ד' ויגיע ט' או כמו י"ו יצורף לדמיונו י"ו ודמיון רביעיתו ד' ויגיע ל"ו או יצורף אל ד' שלשה דמיוניו והוא י"ב ויגיע י"ו או יחוסר מי"ו שלשה רביעיו שההוא י"ב ויגיע ד'
- ↑ marg. א"ב סבת זה כי לא יצאו אחדים באחרית כי אם מהכאת אחדי' באחדים כי מהכאת עשרות או מאות ושאר המדרגות לא יצאו אחדים וקרה שמהכאת האחדים עד ט' בעצמם היו האחדים הנשארים א' ד' ה' ו' ט' והנה תמצא שמהכאת האמצעי אשר במדרגת האחדי' שהוא ה' בעצמו נשאר ה' אח"כ הנשאר מהפאות הרחוקות מהאמצעי מרחק שוה הנה ישוו אחדי גדריהם כי אחדי פ"א שהוא מרבע ט' ישוה לאחד שהוא אחדי גדר א' וט' וא' רחוקים בשוה מן החמשה וכן אחדי ס"ד וד' למספרי ח' וב' ואחדי מ"ט וט' למספרי ז' וג' ואחדי ל"ו וי"ו למספרי ו' וד'
- ↑ marg. א"ב צ"ע בבחינה זו פי' במאזניהם
- ↑ marg. א"ב ר"ל מספר מדרגתו זוג בהתחיל מן האחד כמו ג' וי' שאינם מששים
- ↑ marg. כמו שתמצא ט"ו שהוא המששה השלישי הנה הוא המשלש החמישי וזה בהתחיל מן האחד
- ↑ marg.א"ב הם מספרי א'ד'ז'י' הנזכרי' למעלה שהתוספת ג' ג' ומה שבין שני מספרי א' ד' או ד' ז' או ז' י' הוא שנים על משך המספרי' הטבעיי'
- ↑ marg.א"ב ר"ל נוסף עליו הכאת חצי צלעו במדרגתו מן המחמשים
- ↑ marg. א"ב נראה שהוא בשלשה מדרגות ור"ל שנכה חצי הצלע והוא שנים בשלשה ויהיה ששה והם עם ששה עשר שנים ועשרים
- ↑ marg. א"ב המשל בזה שנרצה לדעת מעקב ג' הנה נכה ג' בד' ויהיה י"ב ונכה י"ב בשנים ויהיו כ"ד נוסיף עליו ג' ויהיה כ"ז יהיה שוה למעקבו וירצה באמרו אח"כ יתוסף אשר לפניו אשר לפני אשר ימשך אליו
- ↑ marg. א"ב משל זה נרצה לדעת המספר הנפרד הראשון שנתרכב ממנו המעקב השלישי שהוא כ"ז הנה תקח מספר מדרגת המעקב הזה מהמספר הראשון שבמעקבים ויהיה ב' וזה חסר ממספר מהמדרגות הראשון שהיה ג' באחד כי כשנתחיל למנות מן האחד יהיו שלשה וכשנתחיל מראשון המספרים המעקבים יהיו שנים וכן תמיד ונגרע ג' שהוא מספר המדרגות השני ממרבע ג' שהוא מספר המדרגות השלישי והוא ט' וישארו ז' והוא הנפרד הראשון אשר ממנו חבור מעקב כ"ז אח"כ נוסיף ב' על המרבע הנזכר ויהיו י"א והוא המספר האחרון משני אלו ממה שביניהם נתרכב כ"ז
- ↑ marg.א"ב ר"ל המספר אשר ימשך אליו והוא הוא המוסיף עליו באחד
- ↑ marg.ר"ל הוהיי הארך שמספר הצלעות שוה והם המרבעים
- ↑ marg. צ"ע כמו שורה אחת
- ↑ marg. א"ב צ"ע ונראה סיומו כיחס ששה אל שלשה ואפשר שהוא כך אשר הוא מותר הארבעה על השלשה כיחס ששה אל שלשה
- ↑ זכרנו
Appendix: Bibliography
Abū al-Ṣalt Umayya b. ‘Abd al-‘Azīz al-Andalusī (b. Andalusia ca. 1068 – d. Tunisia ca. 1134)
– Hebrew translation –
Don Benveniste b. Lavi
Sefer Niqomachus ba-Segulot ha-Mispariyot
1395/1435, Saragossa
Manuscripts:
- 1) Oxford, Bodleian Library MS Heb. d. 3/4 (IMHM: f 22729), ff. 47v-59r (cat. Neub. 2774, 4) (16th century)
- 2) St. Petersburg, Inst. of Oriental Studies of the Russian Academy B 103/1 (IMHM: f 44256), ff. 1r-21v (15th century)
- St.Petersburg103
The transcript is based mainly on manuscript St. Petersburg
Bibliography:
- Langermann, Y. Tzvi. 2001. Studies in Medieval Hebrew Pythagoreanism: Translations and Notes to Nicomachus; Arithmological Texts, Micrologus IX, pp. 219–36.
- Lévy, Tony. 1996. L’histoire des nombres amiables: le témoignage des textes hébreux médiévaux, Arabic Sciences and Philosophy 6, pp. 63–87.
![\scriptstyle n=\frac{1}{2}\sdot\left[\left(n-m\right)+\left(n+m\right)\right]](/mediawiki/images/math/9/c/5/9c5220dd42729dd7ceda90eaea5e82a4.png)
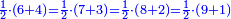
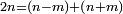
![\scriptstyle\frac{1}{2}\sdot n=\frac{1}{4}\sdot\left[\left(n-m\right)+\left(n+m\right)\right]](/mediawiki/images/math/c/2/3/c2368c2169aef3afd44bbb68b1e44583.png)
![\scriptstyle n^2=\left[\left(n-1\right)\sdot\left(n+1\right)\right]+1](/mediawiki/images/math/b/4/2/b4260f5ae5efcbfb8adcd9588ceef33d.png)
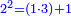
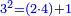

![\scriptstyle n^2-\left[\left(n-m\right)\sdot\left(n+m\right)\right]=m^2](/mediawiki/images/math/c/2/5/c2534a5fa0fa489e9308e83f076f2683.png)
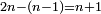
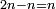

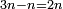
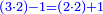
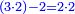
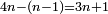
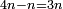
![\scriptstyle\left(m\sdot n\right)-\left(n-1\right)=\left[\left(m-1\right)\sdot n\right]+1](/mediawiki/images/math/8/3/3/833296d312ba1d862ea7a11f65aad7da.png)

![\scriptstyle n^2-\left(n-1\right)=\left[n\sdot\left(n-1\right)\right]+1](/mediawiki/images/math/2/e/8/2e8f3fd7e6862939836d7c2887fbce6c.png)
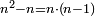
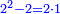
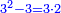
![\scriptstyle n^2=\left[\left(n+1\right)\sdot\left(n-1\right)\right]+1](/mediawiki/images/math/2/2/8/228c62228f173dbb0f8acb2b2842da93.png)
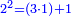
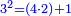
![\scriptstyle\left[n\sdot\left(n-1\right)\right]-\left(n-1\right)=\left(n-1\right)^2](/mediawiki/images/math/f/c/9/fc99bf75bc39bf4caf1eca2000490eb0.png)

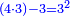
![\scriptstyle\left[n\sdot\left(n+1\right)\right]-n=n^2](/mediawiki/images/math/a/3/e/a3e2be961c71b33740642fb53406703e.png)
 ?
?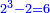
![\scriptstyle n^3-n=\left[n\sdot\left(n+1\right)\right]\sdot\left(n-1\right)](/mediawiki/images/math/a/e/a/aea71daeaade7bda613128faac5a3607.png)
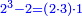
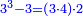
![\scriptstyle n^4-n=\left[n^2+\left(n+1\right)\right]\sdot\left[n\sdot\left(n-1\right)\right]](/mediawiki/images/math/e/f/8/ef8676530f1fd60dcbb1654cced64d45.png)

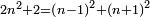
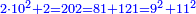


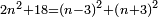
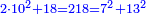



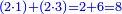
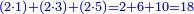
![\scriptstyle2n^2+4=\left[\left(n-2\right)\sdot\left(n-1\right)\right]+\left[\left(n+1\right)\sdot\left(n+2\right)\right]](/mediawiki/images/math/e/d/f/edfd91ae19ebdf94a27e1e45c7240830.png)
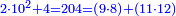
![\scriptstyle2n^2+12=\left[\left(n-2\right)\sdot\left(n-3\right)\right]+\left[\left(n+2\right)\sdot\left(n+3\right)\right]](/mediawiki/images/math/4/d/7/4d75349b28ec77646421533f1c00e0bf.png)
![\scriptstyle2n^2+24=\left[\left(n-3\right)\sdot\left(n-4\right)\right]+\left[\left(n+3\right)\sdot\left(n+4\right)\right]](/mediawiki/images/math/1/e/f/1ef27c8af44c80eb913eec6504ba74c5.png)
![\scriptstyle2n^2+40=\left[\left(n-4\right)\sdot\left(n-5\right)\right]+\left[\left(n+4\right)\sdot\left(n+5\right)\right]](/mediawiki/images/math/d/5/1/d514aa0db6374f000dabf24a08200223.png)


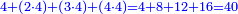
![\scriptstyle2n^2+6=\left[\left(n-1\right)\sdot\left(n-3\right)\right]+\left[\left(n+1\right)\sdot\left(n+3\right)\right]](/mediawiki/images/math/b/6/d/b6d08bfdd80a9ba77cd006f9e9f93d37.png)
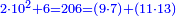
![\scriptstyle2n^2+8=\left[\left(n-1\right)\sdot\left(n-4\right)\right]+\left[\left(n+1\right)\sdot\left(n+4\right)\right]](/mediawiki/images/math/f/d/7/fd72005222fde5847684b4b2d41e0f55.png)
![\scriptstyle2n^2+16=\left[\left(n-2\right)\sdot\left(n-4\right)\right]+\left[\left(n+2\right)\sdot\left(n+4\right)\right]](/mediawiki/images/math/f/f/d/ffd94c709532f4cb997a2fefb55abeab.png)

![\scriptstyle2n^2+20=\left[\left(n-2\right)\sdot\left(n-5\right)\right]+\left[\left(n+2\right)\sdot\left(n+5\right)\right]](/mediawiki/images/math/7/7/e/77e76be56b0d6e6d35b89dfe0d403dbb.png)
![\scriptstyle2n^2+24=\left[\left(n-2\right)\sdot\left(n-6\right)\right]+\left[\left(n+2\right)\sdot\left(n+6\right)\right]](/mediawiki/images/math/6/b/0/6b0255d6e48cb53e70e023418c82b6bf.png)
![\scriptstyle2n^2+30=\left[\left(n-3\right)\sdot\left(n-5\right)\right]+\left[\left(n+3\right)\sdot\left(n+5\right)\right]](/mediawiki/images/math/a/f/6/af6f65bbc997e6a87c8a890a4666eec6.png)
![\scriptstyle2n^2+36=\left[\left(n-3\right)\sdot\left(n-6\right)\right]+\left[\left(n+3\right)\sdot\left(n+6\right)\right]](/mediawiki/images/math/2/b/e/2beba5a6587a3ce96972411b8d33f158.png)
![\scriptstyle2n^2+42=\left[\left(n-3\right)\sdot\left(n-7\right)\right]+\left[\left(n+3\right)\sdot\left(n+7\right)\right]](/mediawiki/images/math/4/b/5/4b5277e92c37684df5b91e14b8a7d025.png)
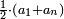
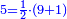
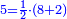
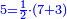
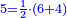
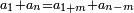

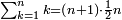
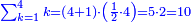
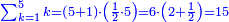
![\scriptstyle\sum_{k=m}^n k=\left(n+m\right)\sdot\left[\frac{1}{2}\sdot\left(n-m+1\right)\right]](/mediawiki/images/math/e/5/a/e5adf0dbee789bd2579afcbe7223033d.png)
![\scriptstyle\sum_{k=m}^n k=\left[\frac{1}{2}\sdot\left(n+m\right)\right]\sdot\left(n-m+1\right)](/mediawiki/images/math/2/0/b/20bebeed8b2d77ce7060b159599d3005.png)
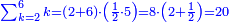
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle\sum_{k=2}^6 k=2+3+4+5+6&\scriptstyle=\left[\frac{1}{2}\sdot\left(2+6\right)\right]\sdot5\\&\scriptstyle=\left(\frac{1}{2}\sdot8\right)\sdot5=4\sdot5=20\\\end{align}}}](/mediawiki/images/math/b/5/0/b50b1ddc006e853205d60f33b2e7fb2c.png)
![\scriptstyle a_n=\left[\left(n-1\right)\sdot d\right]+a_1](/mediawiki/images/math/b/c/f/bcf0c1893f245550c7758eb5f74d4294.png)
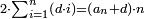
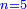 , starting from 4
, starting from 4 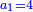 , between every two numbers there are three, so that the excess is four
, between every two numbers there are three, so that the excess is four  , which is the last of them and how much is their sum?
, which is the last of them and how much is their sum?![\scriptstyle{\color{blue}{a_5=\left[\left(5-1\right)\sdot4\right]+4=\left(4\sdot4\right)+4=16+4=20}}](/mediawiki/images/math/d/f/e/dfe4ff338b04a43dffeb392daeb1dbb3.png)
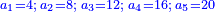
![\scriptstyle{\color{blue}{\sum_{i=1}^5 4i=\frac{1}{2}\sdot\left[\left(20+4\right)\sdot5\right]=\frac{1}{2}\sdot\left(24\sdot5\right)=\frac{1}{2}\sdot120}}](/mediawiki/images/math/b/1/1/b11f9c0c1e45e6acb4bc1ddc924a1cf3.png)
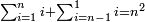
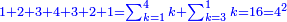

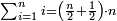
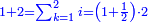

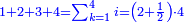

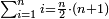
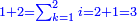
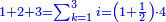

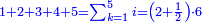
![\scriptstyle\sum_{i=1}^n 2i=\left(1+\frac{1}{n}\right)\sdot\left[\sum_{i=1}^n \left(2i-1\right)\right]](/mediawiki/images/math/c/5/4/c54fadd584df3bbd37e71e4752e5f2bc.png)
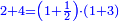
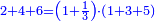
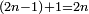 ]
]![\scriptstyle\left[\left(2n-1\right)+1\right]+1=2n+1=\left[2\sdot\left(n+1\right)\right]-1](/mediawiki/images/math/e/a/8/ea8c6a3305842a0ca92cfcea6917294b.png) ]
]![\scriptstyle\left[\left[\left(2n-1\right)+1\right]+1\right]+1=2\sdot\left(n+1\right)](/mediawiki/images/math/3/0/6/306cf0f0b2597dbed70ceb2d26365cf3.png)
![\scriptstyle\left[\left[2\sdot\left(n+1\right)\right]-1\right]-\left(2n-1\right)=2](/mediawiki/images/math/d/c/3/dc3c24da72a3abf4da33ea40880faed4.png)
![\scriptstyle\left[2\sdot\left(n+1\right)\right]-2n=2](/mediawiki/images/math/8/0/b/80b81448159f33d9f7fc0984a9731879.png)
![\scriptstyle n+\left(n+1\right)=\left(n-m\right)+\left[\left(n+1\right)+m\right]](/mediawiki/images/math/1/4/a/14a9c5983a5bc641d63bdb65629ac5c7.png)
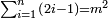


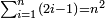
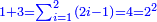
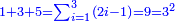
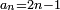
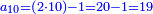
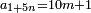


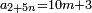
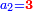

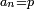 , when extracting from it the interval, whose value is that number, its end
, when extracting from it the interval, whose value is that number, its end  is a composite number that consists of deficient primes.
is a composite number that consists of deficient primes.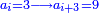 , which is composite.
, which is composite.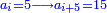 , is composite.
, is composite.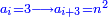 , but afterwards not
, but afterwards not 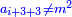
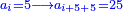 , but afterwards not
, but afterwards not 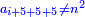
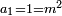 , has a root, which is nine
, has a root, which is nine 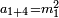 , then the eighth after the first that has a root
, then the eighth after the first that has a root 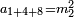 , then the twelfth after the second that has a root
, then the twelfth after the second that has a root  , then the sixteenth after the third that has a root
, then the sixteenth after the third that has a root 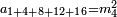 , and so on by adding four
, and so on by adding four 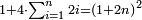

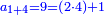

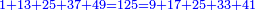 ]
]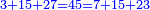
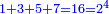 ]
] ]
]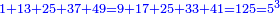 ]
] ]
]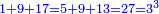 ]
] ; and:
; and: 

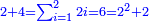
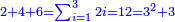
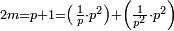
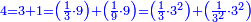
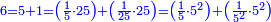
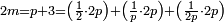
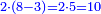
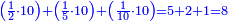
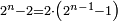 → even-times-odd number
→ even-times-odd number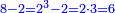
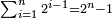 ]
]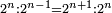
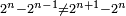



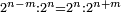
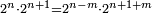

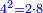

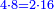

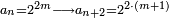
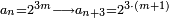

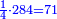
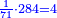
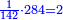
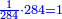
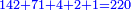




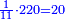




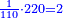

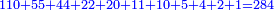
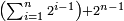 ] and the result of the subtraction [
] and the result of the subtraction [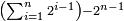 ] are prime numbers.
] are prime numbers.![\scriptstyle a=\left[\left(\sum_{i=1}^n 2^{i-1}\right)+2^{n-1}\right]\sdot\left[\left(\sum_{i=1}^n 2^{i-1}\right)-2^{n-1}\right]\sdot2^{n-1}](/mediawiki/images/math/f/1/a/f1a16a24281539daa583a1502c8fc8f6.png)
![\begin{align}\scriptstyle b&\scriptstyle=\left[\left[\left(\sum_{i=1}^n 2^{i-1}\right)+2^{n-1}\right]\sdot\left[\left(\sum_{i=1}^n 2^{i-1}\right)-2^{n-1}\right]\sdot2^{n-1}\right]\\&\scriptstyle+\left[\left[\left[\left(\sum_{i=1}^n 2^{i-1}\right)+2^{n-1}\right]+\left[\left(\sum_{i=1}^n 2^{i-1}\right)-2^{n-1}\right]\right]\sdot2^{n-1}\right]\\\end{align}](/mediawiki/images/math/c/6/5/c65ed2b72bba8e605ced9a06183a8731.png)

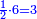
![\scriptstyle\left[2\sdot\left(2n-1\right)\right]+2=2\sdot2n](/mediawiki/images/math/3/2/2/3227327e4c84b9e8b983f435f066a25c.png)
![\scriptstyle\left[2\sdot\left[\left[2\sdot\left(n+1\right)\right]-1\right]\right]-\left[2\sdot\left(2n-1\right)\right]=2\sdot\left[\left[\left[2\sdot\left(n+1\right)\right]-1\right]-\left(2n-1\right)\right]](/mediawiki/images/math/f/f/8/ff8ec7a8ad46e977c80d3201c9f73737.png)
![\scriptstyle\left[2\sdot\left[\left[2\sdot\left(n+1\right)\right]-1\right]\right]-\left[2\sdot\left(2n-1\right)\right]=4](/mediawiki/images/math/7/f/c/7fc2945c117ff0122630cb2b620ad860.png)


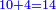
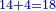

 are even-times-odd numbers divisible by 3]
are even-times-odd numbers divisible by 3] are even-times-odd numbers divisible by 5]
are even-times-odd numbers divisible by 5] are even-times-odd numbers divisible by 7]
are even-times-odd numbers divisible by 7]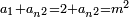
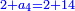
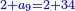
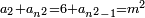

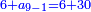

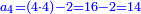
![\scriptstyle n=\frac{\left[2\sdot\left(2n-1\right)\right]+2}{4}=\frac{a_n+2}{4}](/mediawiki/images/math/2/e/a/2ea0a5d7ff15c8af9b5580a5d69e0c0d.png)
![\scriptstyle2n^2=\sum_{i=1}^n \left[2\sdot\left(2i-1\right)\right]](/mediawiki/images/math/3/6/0/3608fbd45e4ae3eb1ccea8b14b38c1a3.png)
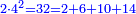
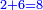 ], and its term is already known, also the cube of the cube and so on [??]
], and its term is already known, also the cube of the cube and so on [??]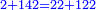

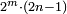
![\scriptstyle\left[2\sdot\left(2n-1\right)\right]^2](/mediawiki/images/math/c/4/a/c4a4088a09b73950cbbc774e7d145e48.png) :
: , which is the root of the first square.
, which is the root of the first square.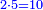 , which is the root of the second square.
, which is the root of the second square.![\scriptstyle\left[2^m\sdot\left[\left[2\sdot\left(n+1\right)\right]-1\right]\right]-\left[2^m\sdot\left(2n-1\right)\right]=2^{m+1}](/mediawiki/images/math/3/0/f/30f8f2ec51a1b51f8404d840fc7f7e5c.png)

![\scriptstyle\left[4\sdot\left[\left[2\sdot\left(n+1\right)\right]-1\right]\right]-\left[4\sdot\left(2n-1\right)\right]](/mediawiki/images/math/2/b/9/2b952ce8d9339a0c1d807877dc17afd5.png)
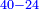
![\scriptstyle\left[8\sdot\left[\left[2\sdot\left(n+1\right)\right]-1\right]\right]-\left[8\sdot\left(2n-1\right)\right]](/mediawiki/images/math/0/f/3/0f3feba94c8998496e3663e08424efe3.png)





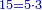

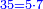
 and
and 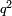 are relatively prime]
are relatively prime]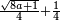 is an even-times-even number; and
is an even-times-even number; and ![\scriptstyle\left(\frac{\sqrt{8a+1}}{4}+\frac{1}{4}\right)\sdot\left[\left[2\sdot\left(\frac{\sqrt{8a+1}}{4}+\frac{1}{4}\right)\right]-1\right]=a](/mediawiki/images/math/2/4/b/24b671f1389b9766f76ad260efe60dd7.png) ]
]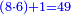
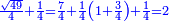 is an even-times-even number
is an even-times-even number![\scriptstyle{\color{blue}{2\sdot\left[\left(2\sdot2\right)-1\right]=6}}](/mediawiki/images/math/7/5/1/7516d3a4e7c3f3303dc7765f59ac0735.png)
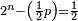 →
→ 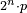 is a perfect number]
is a perfect number]
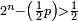 →
→ 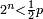 →
→  [
[ [
[
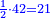

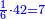
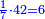
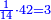
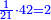
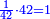
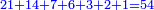
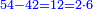
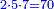
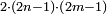 is superabundant
is superabundant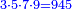

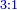
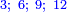

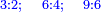

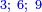
 ]: The second line is in the double ratio to the first line.
]: The second line is in the double ratio to the first line. ]: The third [line] is in the triple ratio.
]: The third [line] is in the triple ratio. ]: The third line is in the sesquialter ratio to the second line.
]: The third line is in the sesquialter ratio to the second line. ]: The fourth [line] is in the sesquitertian ratio to the third [line].
]: The fourth [line] is in the sesquitertian ratio to the third [line].  ]: The fifth [line] is in the sesquiquartan ratio to the fourth [line].
]: The fifth [line] is in the sesquiquartan ratio to the fourth [line]. ]: The excesses of the second line over the first line differ by number, but not differ by ratio.
]: The excesses of the second line over the first line differ by number, but not differ by ratio.

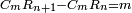 ]
] ]: When the third is related to the first in every sequence they are found by the order of the even numbers.
]: When the third is related to the first in every sequence they are found by the order of the even numbers.
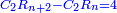

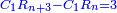

 ]
] [?]
[?]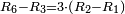 [?]
[?]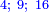
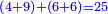
![\scriptstyle\left[2\sdot\left[n^2+\left(n+1\right)^2\right]\right]-1=m^2](/mediawiki/images/math/1/b/5/1b5cf8fdc120fa6c33050cf4008a04b6.png)
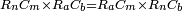
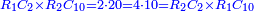
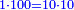

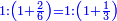
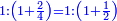
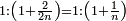 ]
]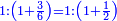
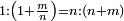 ]
]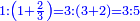 ]
]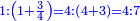 ]
] :
: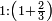
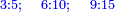
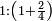 , which is not absolute
, which is not absolute
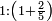
![\scriptstyle\left[1:\left(1+\frac{2}{3}\right)\right];\quad\left[1:\left(1+\frac{2}{4}\right)\right];\quad\left[1:\left(1+\frac{2}{5}\right)\right]](/mediawiki/images/math/8/9/3/8936d012e4df99bde083b4893902cbda.png)
![\scriptstyle\left[1:\left(1+\frac{2}{2n-1}\right)\right]:\left[1:\left(1+\frac{2}{2n+1}\right)\right]](/mediawiki/images/math/8/4/5/8451e01f8feb2bebdca675724dae4f88.png) ]
]
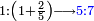
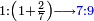
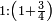


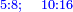
![\scriptstyle\left[1:\left(1+\frac{3}{4}\right)\right];\quad\left[1:\left(1+\frac{3}{5}\right)\right];\quad\left[1:\left(1+\frac{3}{6}\right)\right]](/mediawiki/images/math/7/4/2/7429bd3b015728006a28b251133d1278.png) ]
]
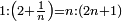
![\scriptstyle{\color{blue}{1:\left(2+\frac{1}{2}\right)=2:\left[\left(2\sdot2\right)+1\right]=2:5}}](/mediawiki/images/math/2/b/1/2b15e422eefe9740ec57253877f5babb.png) ]
]![\scriptstyle{\color{blue}{1:\left(2+\frac{1}{3}\right)=3:\left[\left(2\sdot3\right)+1\right]=3:7}}](/mediawiki/images/math/7/8/b/78b8889b85a9e312e5e794a0afa584f2.png) ]
]![\scriptstyle{\color{blue}{1:\left(2+\frac{1}{4}\right)=4:\left[\left(2\sdot4\right)+1\right]=4:9}}](/mediawiki/images/math/4/8/d/48dc239e9cc60a3aeaeb5d6b389911b7.png) ]
]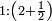 ]:
]:
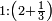 :
:
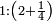 :
: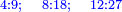
![\scriptstyle\left[1:\left(2+\frac{1}{2}\right)\right];\quad\left[1:\left(2+\frac{1}{3}\right)\right];\quad\left[1:\left(2+\frac{1}{4}\right)\right]](/mediawiki/images/math/0/a/9/0a96ac733bf4e6287b99e10dbe90968b.png) ]
]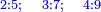 and so on in this way.
and so on in this way.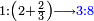
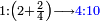

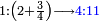
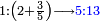
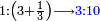
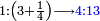



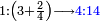
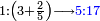

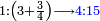
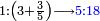

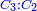 ]:The superparticular ratio is resulted, as already known, from the third and second columns.
]:The superparticular ratio is resulted, as already known, from the third and second columns.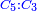 ]: The superbitertian ratio from the fifth and the third columns.
]: The superbitertian ratio from the fifth and the third columns.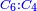 ]: The superbiquartan ratio from the sixth and the fourth columns.
]: The superbiquartan ratio from the sixth and the fourth columns. ]: The superbiquintan ratio from the seventh and the fifth columns.
]: The superbiquintan ratio from the seventh and the fifth columns.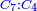 ]: From the seventh and the fourth columns = the supertriquartan ratio.
]: From the seventh and the fourth columns = the supertriquartan ratio.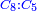 ]: From the eighth and the fifth columns - the supertriquintan ratio.
]: From the eighth and the fifth columns - the supertriquintan ratio.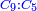 ]: From the ninth and the fifth columns - the superquadriquintan ratio.
]: From the ninth and the fifth columns - the superquadriquintan ratio.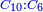 ]: From the tenth and the sixth columns - the superquadrisextan ratio.
]: From the tenth and the sixth columns - the superquadrisextan ratio.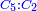 ]: The first of them, the double sesquialter ratio, from the fifth and the second columns.
]: The first of them, the double sesquialter ratio, from the fifth and the second columns.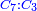 ]: The second, the double sesquitertian ratio, from the seventh and the third columns.
]: The second, the double sesquitertian ratio, from the seventh and the third columns.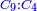 ]: The third, the double sesquiquartan ratio, from the ninth and the fourth columns.
]: The third, the double sesquiquartan ratio, from the ninth and the fourth columns.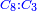 ]: The double superbitertian [ratio], from the eighth and the third [columns].
]: The double superbitertian [ratio], from the eighth and the third [columns]. ]: The double superbiquartan [ratio], from the tenth and the fourth [columns].
]: The double superbiquartan [ratio], from the tenth and the fourth [columns]. ]
]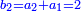 ]
]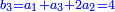 ]
]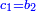 ]
] ]: what is on the second row is in the double ratio.
]: what is on the second row is in the double ratio. ]: what is on the third row is in the triple ratio.
]: what is on the third row is in the triple ratio. ]: what is on the fourth row is in the quadruple ratio.
]: what is on the fourth row is in the quadruple ratio.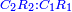 ]: what is in the second rubric of the second row to what is in the first rubric is in the quadruple ratio.
]: what is in the second rubric of the second row to what is in the first rubric is in the quadruple ratio.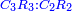 ]: what is in the third rubric to what is in the second rubric is in the double sesquiquartan ratio.
]: what is in the third rubric to what is in the second rubric is in the double sesquiquartan ratio.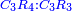 ]: what is in the fourth rubric to what is in the third rubric is in the superseptinona ratio.
]: what is in the fourth rubric to what is in the third rubric is in the superseptinona ratio.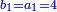 ]
]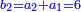 ]
]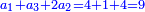 ]
]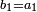 ].
].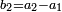 ].
].![\scriptstyle b_3=a_3-\left[2\left(a_2-a_1\right)+a_1\right]=a_3-\left(2b_2+b_1\right)](/mediawiki/images/math/2/8/2/28241de1fdee9a0aaf58885a6fab520b.png) ].
].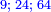
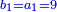
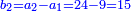
![\scriptstyle{\color{blue}{b_3=a_3-\left[2\left(a_2-a_1\right)+a_1\right]=64-\left[\left(2\sdot15\right)+9\right]=25}}](/mediawiki/images/math/7/1/8/718b329d3f4206cbf8afbf69283299e8.png)
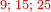 ] in the superbitertian ratio.
] in the superbitertian ratio.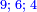 , which are successive numbers in the sesquialter ratio.
, which are successive numbers in the sesquialter ratio.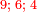 ] by this procedure, and the result are
] by this procedure, and the result are  , which are in the double ratio.
, which are in the double ratio. , restored to equality.
, restored to equality.
 ] and the sesquiseptan ratio [
] and the sesquiseptan ratio [ ] is the sesquitertian ratio [
] is the sesquitertian ratio [ ]
]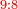 ] is the superbiseptan ratio [
] is the superbiseptan ratio [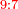 ]
] ] is the sesquiquartan ratio [
] is the sesquiquartan ratio [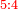 ]
]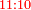 ] is the superbinona ratio [
] is the superbinona ratio [ ]
] ] is the sesquiquintan ratio [
] is the sesquiquintan ratio [ ]
] ] is the superbiundecima ratio [
] is the superbiundecima ratio [ ]
] ] is the sesquisextan ratio [
] is the sesquisextan ratio [ ] is the superbitertdecima ratio [
] is the superbitertdecima ratio [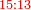 ]
]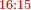 ] is the sesquiseptian ratio [
] is the sesquiseptian ratio [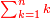 ].
].![\scriptstyle{\color{red}{\sum_{k=1}^n k=\frac{1}{2}\sdot\left[n\sdot\left(n+1\right)\right]}}](/mediawiki/images/math/d/a/3/da36748df8ff59ff88435b913e6c55d2.png) ]
]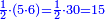 is the fifth triangle [in potential].
is the fifth triangle [in potential].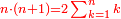 ].
]. → its side is five.
→ its side is five.
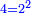
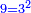
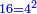
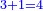 which is the first of the square numbers.
which is the first of the square numbers. which is the second square number.
which is the second square number.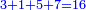 which is the third square number.
which is the third square number.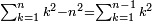 .
.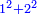 exceeds the square of two
exceeds the square of two 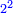 by the square of one
by the square of one 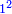 .
.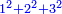 exceeds the square of three
exceeds the square of three 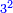 by the sum of the two squares of one and two
by the sum of the two squares of one and two 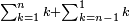 .
.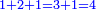 which is the first square number.
which is the first square number. which is the second square number.
which is the second square number. which is the third square of the square numbers.
which is the third square of the square numbers.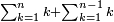 .
.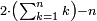 .
.![\scriptstyle n^2+m^2+\left[2\sdot\left(n\sdot m\right)\right]](/mediawiki/images/math/8/2/8/8287d72fc47eefd97b148843d3c9ede1.png) .
.![\scriptstyle{\color{blue}{4+9+\left[2\sdot\left(2\sdot3\right)\right]=25}}](/mediawiki/images/math/0/9/3/0937e73c233767419967902c47ace1a2.png)
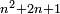 , or when it is added to its same and a quarter of its same
, or when it is added to its same and a quarter of its same 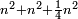 , or thrice its same
, or thrice its same 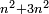 , or when its three quarters are subtracted from it
, or when its three quarters are subtracted from it 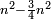 - the results are squares.
- the results are squares.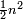 or double
or double 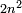 are squares.
are squares. ]: The first of them is the five, which is composed as this:
]: The first of them is the five, which is composed as this: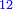 ]: The second pentagonal is that whose side is the second number, which is three, so the pentagonal formed from it is twelve, like this:
]: The second pentagonal is that whose side is the second number, which is three, so the pentagonal formed from it is twelve, like this: ]: The third number, which is four, the pentagonal that is formed from it is twenty two, like this:
]: The third number, which is four, the pentagonal that is formed from it is twenty two, like this: with the one
with the one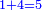 which is the first pentagonal number.
which is the first pentagonal number.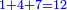 which is the second pentagonal number.
which is the second pentagonal number. which is the third pentagonal number.
which is the third pentagonal number.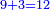 .
.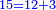 = the second pentagonal number + the first triangular number.
= the second pentagonal number + the first triangular number. = the second hexagonal number + the first triangular number.
= the second hexagonal number + the first triangular number. ] = the first pentagonal number.
] = the first pentagonal number. ] = the third pentagonal number.
] = the third pentagonal number. ]
]
 ] = the first hexagonal number.
] = the first hexagonal number. ] = the second hexagonal number.
] = the second hexagonal number.![\scriptstyle{\color{red}{\left(n+1\right)^2+\left[\left(n+1\right)\sdot n\left(1+\frac{1}{2}\right)\right]}}](/mediawiki/images/math/1/2/a/12a39626c58d1b0225254376b46f5fda.png) ]
]
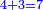 ] = the first heptagonal number.
] = the first heptagonal number. ] = the second heptagonal number.
] = the second heptagonal number. = the first hexagonal number = the second triangular number.
= the first hexagonal number = the second triangular number.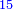 = the second hexagonal number = the fourth triangular number.
= the second hexagonal number = the fourth triangular number. = the third hexagonal number = the sixth triangular number.
= the third hexagonal number = the sixth triangular number.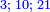 , which are triangles, but not hexagonal numbers.
, which are triangles, but not hexagonal numbers.![\scriptstyle{\color{red}{\frac{1}{2}\sdot\left[\left[\left[3\sdot\left(n-1\right)\right]+2\right]\sdot n\right]}}](/mediawiki/images/math/9/3/e/93e6cc3c933e850b4375b351b8849fde.png) ]
]![\scriptstyle{\color{blue}{\frac{1}{2}\sdot\left[\left[\left(3\sdot3\right)+2\right]\sdot4\right]=\frac{1}{2}\sdot\left[\left(9+2\right)\sdot4\right]=\frac{1}{2}\sdot\left(11\sdot4\right)=\frac{1}{2}\sdot44=22}}](/mediawiki/images/math/a/d/1/ad1bb1cb563e2d2c763ba15700b8a782.png)
![\scriptstyle{\color{red}{n^2+\left[\left(\frac{1}{2}\sdot n\right)\sdot\left(n-1\right)\right]}}](/mediawiki/images/math/1/1/0/1104c405e7db41eab68b7cbee58a5c3a.png) ]
]![\scriptstyle{\color{blue}{\left(4\sdot4\right)+\left[\left(\frac{1}{2}\sdot4\right)\sdot3\right]=16+\left(2\sdot3\right)=22}}](/mediawiki/images/math/1/7/a/17a6757180909a40697a627d465b9a87.png)
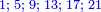
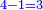 a prime number, appropriate for the procedure.
a prime number, appropriate for the procedure. 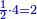
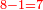 prime numbe, appropriate for the requested [procedure]
prime numbe, appropriate for the requested [procedure]
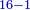 composite number, not appropriate for the procedure
composite number, not appropriate for the procedure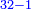 prime number, appropriate for the procedure
prime number, appropriate for the procedure
 .
.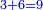 the second square.
the second square.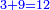 the second pentagon.
the second pentagon.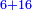 the third pentagon.
the third pentagon. .
.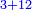 the second hexagon.
the second hexagon. = The first pyramid.
= The first pyramid.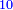 = The second pyramid of this type.
= The second pyramid of this type.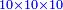 - called cube.
- called cube.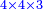




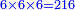
![\scriptstyle\left[n\sdot\left(n+1\right)\sdot\left(n-1\right)\right]+n=n^3](/mediawiki/images/math/a/9/9/a996064cf0852665f551c754512475b1.png)
 which is a cube.
which is a cube.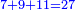 which is the third cube.
which is the third cube.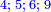 are always recurring in the units of their cubes, this is the instruction of the units of the root of the cube.
are always recurring in the units of their cubes, this is the instruction of the units of the root of the cube.

![\scriptstyle{\color{red}{\left(n+1\right)^3-n^3=\left[n\sdot\left(n+1\right)\sdot3\right]+1}}](/mediawiki/images/math/3/e/6/3e63601006ddff16294ac3b53a745230.png) ]
]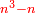 divisible by 6]
divisible by 6] divisible by
divisible by  ]
]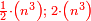 are not cubes]
are not cubes]![\scriptstyle{\color{red}{n^3+1+6\sdot\left[\frac{1}{2}\sdot\left[n\sdot\left(n+1\right)\right]\right]=\left(n+1\right)^3}}](/mediawiki/images/math/7/f/c/7fcce4c6a0816afdc329bdc45d0a7d71.png) ]
]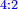 double ratio
double ratio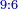 sesquialter ratio
sesquialter ratio sesquitertian ratio
sesquitertian ratio
 sesquialter ratio
sesquialter ratio



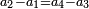
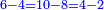
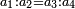
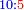
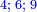
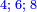
 sesquialter ratio
sesquialter ratio sesquitertian ratio
sesquitertian ratio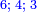 , and it is called harmonic, since the benefit from the observation of this proportion is fulfilled in the art of composition, which is music, as will be known in its place.
, and it is called harmonic, since the benefit from the observation of this proportion is fulfilled in the art of composition, which is music, as will be known in its place.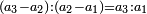
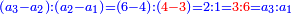


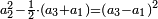

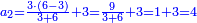
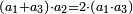
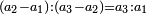
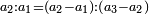
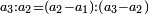
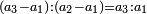
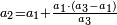
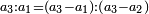
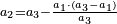
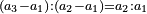
![\scriptstyle\left(\frac{1}{2}\sdot a_1\right)+\sqrt{\left(\frac{1}{2}\sdot a_1\right)^2+\left[\left(a_3-a_1\right)\sdot a_1\right]}](/mediawiki/images/math/e/a/d/eada53cd27cf9472b216bb51d80d9592.png)
![\scriptstyle\left(\frac{1}{2}\sdot a_3\right)+\sqrt{\left(\frac{1}{2}\sdot a_3\right)^2-\left[\left(a_3-a_1\right)\sdot a_1\right]}](/mediawiki/images/math/0/9/f/09fe8930525048d5491e216bfbf3c630.png)
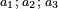 ]
]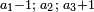 ] and it is the sixth proportion.
] and it is the sixth proportion.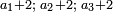 ] the ninth proportion is resulted.
] the ninth proportion is resulted.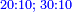
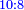

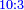
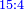
![\scriptstyle\left(1+\frac{1}{n}\right):1=\left[n+\left(\frac{1}{n}\sdot n\right)\right]:n](/mediawiki/images/math/2/9/4/294dfe97e860deae2175a807a062caf2.png)
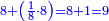
 is the sesquioctavian ratio.
is the sesquioctavian ratio.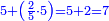
 is the superbiquintan ratio.
is the superbiquintan ratio.![\scriptstyle\left(m+\frac{a}{n}\right):1=\left[\left(m\sdot n\right)+\left(\frac{a}{n}\sdot n\right)\right]:n](/mediawiki/images/math/0/3/c/03cb195620c16cccc85b497ce6b8a366.png)
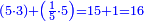 which is the greater.
which is the greater.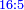 is the triple sesquiquintan ratio.
is the triple sesquiquintan ratio. 
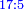 is the triple superbiquintan ratio.
is the triple superbiquintan ratio. 

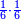
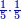
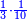

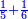
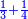

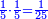
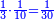
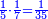
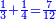
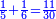
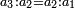 .
.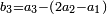 .
.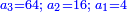
![\scriptstyle{\color{blue}{b_3=a_3-\left(2a_2-a_1\right)=64-\left[\left(2\sdot16\right)-4\right]=64-28=36}}](/mediawiki/images/math/e/d/b/edbc2d3be9a7645d778227d61c7c794f.png)
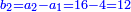
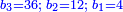 are in the triple ratio.
are in the triple ratio.![\scriptstyle{\color{blue}{c_3=b_3-\left[2b_2-b_1\right]=36-\left[\left(2\sdot12\right)-4\right]=36-20=16}}](/mediawiki/images/math/a/3/8/a381fb3334579961721207bddab4ae86.png)
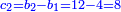
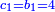
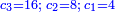 are in the double ratio.
are in the double ratio.![\scriptstyle{\color{blue}{d_3=c_3-\left[2c_2-c_1\right]=16-\left[\left(2\sdot8\right)-4\right]=16-12}}](/mediawiki/images/math/d/1/0/d10ebaae8b410047b51d0823d05b6b68.png)
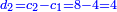
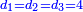
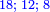
![\scriptstyle{\color{blue}{b_1=a_3-\left[2a_2-a_1\right]=18-\left[\left(2\sdot12\right)-8\right]=18-16=2}}](/mediawiki/images/math/3/f/3/3f342d4f77c4d0edc7a2010a3a55d360.png)
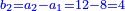
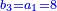
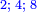 are in the double ratio.
are in the double ratio.![\scriptstyle{\color{blue}{c_3=b_3-\left[2b_2-b_1\right]=8-\left[\left(2\sdot4\right)-2\right]=8-6=2}}](/mediawiki/images/math/1/a/a/1aacc8a0f3da7e9651e4a4cf6b9858ac.png)

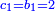

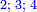 succeeding one by one
succeeding one by one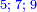 succeeding two by two
succeeding two by two superbiseptian ratio
superbiseptian ratio and
and 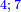 and
and 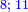
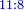 is the same and a quarter and an eighth.
is the same and a quarter and an eighth. is the same and a half and a quarter.
is the same and a half and a quarter. double sesquialter ratio
double sesquialter ratio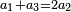 ; and of the discontinuous, it is equal to the sum of the means
; and of the discontinuous, it is equal to the sum of the means 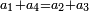 .
. 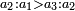 and
and 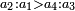
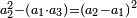 .
.  .
.![\scriptstyle n=\frac{1}{2}\sdot\left[\left(n-1\right)+\left(n+1\right)\right]](/mediawiki/images/math/6/6/a/66a4f5c01b212c7e738a63bbb1276d70.png) .
.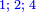 for the continuous.
for the continuous.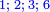 for the discontinuous.
for the discontinuous.

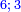 and
and  whose ratio is the same, but their differences are different.
whose ratio is the same, but their differences are different.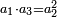 ; and it is equal to the product of one of the means by the other, of the discontinuous
; and it is equal to the product of one of the means by the other, of the discontinuous 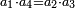 .
. 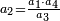 .
.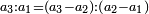
 .
.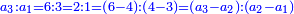
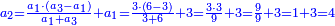
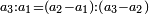
 .
.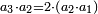 [incorrect for the general case]
[incorrect for the general case]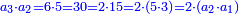
 .
.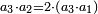 [incorrect for the general case]
[incorrect for the general case]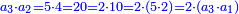
 .
.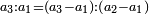
 .
.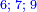 .
.
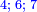 .
.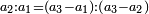
 .
.