Difference between revisions of "ספר ג'יבלי אלמוקבאלא"
(→Algebra) |
|||
| Line 1: | Line 1: | ||
| + | {{#annotpage: author="Maestro Dardi", translator="Mordecai Finzi", city="Mantua", time="unknown-1475", peshat_title="00002029"}} | ||
| + | __TOC__ | ||
| + | <br> | ||
{| | {| | ||
|- | |- | ||
| − | | | + | |These are the signs of the six abbreviated chapters of the book Jīblī al-Mūqabāla, |
| − | |style="text-align:right;"|אלו הם הסימנים מהששה פרקים מקוצרים מספר גבלי אלמוקבאלא | + | |style="text-align:right;"|‫<ref>Paris 194r</ref>אלו הם הסימנים מהששה פרקים מקוצרים מספר גבלי אלמוקבאלא |
|- | |- | ||
| − | | | + | |and of those written and explained by Master Dardi from Pisa, |
|style="text-align:right;"|ומאשר האומן דארדי מפיסא כתב ופירש עליהם | |style="text-align:right;"|ומאשר האומן דארדי מפיסא כתב ופירש עליהם | ||
|- | |- | ||
| − | | | + | |which are 194 in number. |
|style="text-align:right;"|אשר הם במספר קצ"ד | |style="text-align:right;"|אשר הם במספר קצ"ד | ||
|- | |- | ||
| − | | | + | |The names of the quantities that will be spoken of are five, which are: |
|style="text-align:right;"|שמות הכמויות אשר ידובר בהם הם חמשה והם | |style="text-align:right;"|שמות הכמויות אשר ידובר בהם הם חמשה והם | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|מספר או דראמא | + | *number or drama |
| + | |style="text-align:right;"|{{#annot:term|242,1174|dADw}}מספר{{#annotend:dADw}} או {{#annot:term|242,1305|jOOp}}דראמא{{#annotend:jOOp}} | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|דבר או שורש | + | *thing or root |
| + | |style="text-align:right;"|{{#annot:term|133,1309|vEta}}דבר{{#annotend:vEta}} או {{#annot:term|133,1262|oap6}}שורש{{#annotend:oap6}} | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|צינסו | + | *çenso |
| + | |style="text-align:right;"|{{#annot:term|687,1313|TF4O}}צינסו{{#annotend:TF4O}} | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *cube |
| + | |style="text-align:right;"|ו{{#annot:term|688,1828|4mEv}}מעוקב{{#annotend:4mEv}} | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *çenso of çenso |
| + | |style="text-align:right;"|ו{{#annot:term|689|iVFX}}צינסו מצינסו{{#annotend:iVFX}} | ||
|- | |- | ||
| + | |[Their abbreviations]: | ||
| | | | ||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|ובמקום | + | *the letter מ [Mem] for mispar [= number], or 'דר [dr] for drama |
| + | |style="text-align:right;"|ובמקום המספר או הדראמא אנו נשים מ' או דר‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|ובמקום | + | *the letter ד [Dalet] for davar [= thing], or the letter ש [Shin] for shoresh [= root] |
| + | |style="text-align:right;"|ובמקום הדבר או שרש אנחנו נשים ד' או ש‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|ובמקום | + | *the letter צ [Tzaddik] for çenso |
| + | |style="text-align:right;"|ובמקום הצינסו צ‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *'מעו [meʼu] for meʼuqav [= cube] |
| + | |style="text-align:right;"|ובמקום המעוקב מעו‫' | ||
|- | |- | ||
| | | | ||
| + | *'צ' מצ for çenso di çenso | ||
| + | |style="text-align:right;"|ובעד ה{{#annot:term|689|9Mat}}צינסו דצינסו{{#annotend:9Mat}} צ' מצ‫' | ||
| + | |- | ||
| + | |As is seen in the categories from here on. | ||
|style="text-align:right;"|כפי אשר תראה בחלוקות מכאן ולהבא | |style="text-align:right;"|כפי אשר תראה בחלוקות מכאן ולהבא | ||
| + | |- | ||
| + | |First the thing is defined. | ||
| + | |style="text-align:right;"|וראשונה נשים הדבר | ||
|- | |- | ||
| | | | ||
| − | + | == Table of Contents == | |
| + | |||
| + | | | ||
|- | |- | ||
| | | | ||
| Line 111: | Line 132: | ||
| | | | ||
*chapter 16: <math>\scriptstyle bx^2+cx=ax^3</math> | *chapter 16: <math>\scriptstyle bx^2+cx=ax^3</math> | ||
| − | |style="text-align:right;"|פרק י"ו צינסו ודבר למעו' | + | |style="text-align:right;"|פרק י"ו צינסו ודבר למעו‫' |
|- | |- | ||
| | | | ||
| Line 127: | Line 148: | ||
| | | | ||
*chapter 20: <math>\scriptstyle c=\sqrt{ax^2}</math> | *chapter 20: <math>\scriptstyle c=\sqrt{ax^2}</math> | ||
| − | |style="text-align:right;"|פרק כ' מספר לשרש צינ' | + | |style="text-align:right;"|פרק כ' מספר לשרש צינ‫' |
|- | |- | ||
| | | | ||
| Line 135: | Line 156: | ||
| | | | ||
*chapter 22: <math>\scriptstyle c=\sqrt{ax^3}</math> | *chapter 22: <math>\scriptstyle c=\sqrt{ax^3}</math> | ||
| − | |style="text-align:right;"|פרק כ"ב מספר לשרש מעו' | + | |style="text-align:right;"|פרק כ"ב מספר לשרש מעו‫' |
|- | |- | ||
| | | | ||
| Line 143: | Line 164: | ||
| | | | ||
*chapter 24: <math>\scriptstyle c=\sqrt{ax^4}</math> | *chapter 24: <math>\scriptstyle c=\sqrt{ax^4}</math> | ||
| − | |style="text-align:right;"|פרק כ"ד מס' לשרש צינסו מצי' | + | |style="text-align:right;"|פרק כ"ד מס' לשרש צינסו מצי‫' |
|- | |- | ||
| | | | ||
| Line 155: | Line 176: | ||
| | | | ||
*chapter 27: <math>\scriptstyle bx=\sqrt{ax^3}</math> | *chapter 27: <math>\scriptstyle bx=\sqrt{ax^3}</math> | ||
| − | |style="text-align:right;"|פרק כ"ז דבר לשרש מעוק' | + | |style="text-align:right;"|פרק כ"ז דבר לשרש מעוק‫' |
|- | |- | ||
| | | | ||
*chapter 28: <math>\scriptstyle bx=\sqrt{ax^4}</math> | *chapter 28: <math>\scriptstyle bx=\sqrt{ax^4}</math> | ||
| − | |style="text-align:right;"|פרק כ"ח דבר לשרש צינ' מצי' | + | |style="text-align:right;"|פרק כ"ח דבר לשרש צינ' מצי‫' |
|- | |- | ||
| | | | ||
| Line 167: | Line 188: | ||
| | | | ||
*chapter 30: <math>\scriptstyle bx^2=\sqrt{ax^3}</math> | *chapter 30: <math>\scriptstyle bx^2=\sqrt{ax^3}</math> | ||
| − | |style="text-align:right;"|פרק ל' צינסו לשרש מעו' | + | |style="text-align:right;"|פרק ל' צינסו לשרש מעו‫' |
|- | |- | ||
| | | | ||
| Line 183: | Line 204: | ||
| | | | ||
*chapter 34: <math>\scriptstyle bx^3=\sqrt{ax^4}</math> | *chapter 34: <math>\scriptstyle bx^3=\sqrt{ax^4}</math> | ||
| − | |style="text-align:right;"|פרק ל"ד מעו' לשרש צינ' מצי' | + | |style="text-align:right;"|פרק ל"ד מעו' לשרש צינ' מצי‫' |
|- | |- | ||
| | | | ||
*chapter 35: <math>\scriptstyle ax^4=\sqrt{bx^4}</math> | *chapter 35: <math>\scriptstyle ax^4=\sqrt{bx^4}</math> | ||
| − | |style="text-align:right;"|פרק ל"ה צי' מצי' לשרש צי' מצי' | + | |style="text-align:right;"|פרק ל"ה צי' מצי' לשרש צי' מצי‫' |
|- | |- | ||
| | | | ||
*chapter 36: <math>\scriptstyle bx=c+\sqrt{d}</math> | *chapter 36: <math>\scriptstyle bx=c+\sqrt{d}</math> | ||
| − | |style="text-align:right;"|פרק ל"ו דבר למספ' ולשרש מספ' | + | |style="text-align:right;"|פרק ל"ו דבר למספ' ולשרש מספ‫' |
|- | |- | ||
| | | | ||
| Line 199: | Line 220: | ||
| | | | ||
*chapter 38: <math>\scriptstyle ax^2=c+\sqrt{d}</math> | *chapter 38: <math>\scriptstyle ax^2=c+\sqrt{d}</math> | ||
| − | |style="text-align:right;"|פרק ל"ח צי' למספר ולשרש מספ' | + | |style="text-align:right;"|פרק ל"ח צי' למספר ולשרש מספ‫' |
|- | |- | ||
| | | | ||
*chapter 39: <math>\scriptstyle c=ax^2+\sqrt{bx^2}</math> | *chapter 39: <math>\scriptstyle c=ax^2+\sqrt{bx^2}</math> | ||
| − | |style="text-align:right;"|פרק ל"ט מס' לצינ' ולשרש צינ' | + | |style="text-align:right;"|פרק ל"ט מס' לצינ' ולשרש צינ‫' |
|- | |- | ||
| | | | ||
*chapter 40: <math>\scriptstyle ax^3=c+\sqrt{d}</math> | *chapter 40: <math>\scriptstyle ax^3=c+\sqrt{d}</math> | ||
| − | |style="text-align:right;"|פרק מ' מעו' למספ' ולשרש מס' | + | |style="text-align:right;"|פרק מ' מעו' למספ' ולשרש מס‫' |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|פרק מ"א מס' למעו' ולשרש מעו' | + | *chapter 41: <math>\scriptstyle c=ax^3+\sqrt{bx^3}</math> |
| + | |style="text-align:right;"|פרק מ"א מס' למעו' ולשרש מעו‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|פרק מ"ב צי' מצי' למס' ולשרש מס' | + | *chapter 42: <math>\scriptstyle ax^4=c+\sqrt{d}</math> |
| + | |style="text-align:right;"|פרק מ"ב צי' מצי' למס' ולשרש מס‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|פרק מ"ג מספ' לצי' מצי' ולש' צי' מצי' | + | *chapter 43: <math>\scriptstyle c=ax^4+\sqrt{bx^4}</math> |
| + | |style="text-align:right;"|פרק מ"ג מספ' לצי' מצי' ולש' צי' מצי‫' | ||
|- | |- | ||
| | | | ||
| + | *chapter 44: <math>\scriptstyle ax^4+bx^2=c</math> | ||
|style="text-align:right;"|פרק מ"ד צי' מצי' וצינ' למספר | |style="text-align:right;"|פרק מ"ד צי' מצי' וצינ' למספר | ||
|- | |- | ||
| | | | ||
| + | *chapter 45: <math>\scriptstyle bx^2=ax^4+c</math> | ||
|style="text-align:right;"|פרק מ"ה צי' לצי' מצי' ולמספר | |style="text-align:right;"|פרק מ"ה צי' לצי' מצי' ולמספר | ||
|- | |- | ||
| | | | ||
| + | *chapter 46: <math>\scriptstyle ax^4=bx^2+c</math> | ||
|style="text-align:right;"|פרק מ"ו צי' מצי' לצי' ולמספר | |style="text-align:right;"|פרק מ"ו צי' מצי' לצי' ולמספר | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|פרק מ"ז צינסו לצינ' מצי' ולמספר | + | *chapter 47: <math>\scriptstyle bx^2=ax^4+c</math> |
| + | |style="text-align:right;"|‫<ref>Paris 194v</ref>פרק מ"ז צינסו לצינ' מצי' ולמספר | ||
|- | |- | ||
| | | | ||
| + | *chapter 48: <math>\scriptstyle c=\sqrt[3]{bx}</math> | ||
|style="text-align:right;"|פרק מ"ח מספ' לשרש מעו' מדבר | |style="text-align:right;"|פרק מ"ח מספ' לשרש מעו' מדבר | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|פרק מ"ט צינסו לשרש מעו' ממספ' | + | *chapter 49: <math>\scriptstyle ax^2=\sqrt[3]{c}</math> |
| + | |style="text-align:right;"|פרק מ"ט צינסו לשרש מעו' ממספ‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|פרק נ' מספר לשרש מעו' מצי' | + | *chapter 50: <math>\scriptstyle c=\sqrt[3]{ax^2}</math> |
| + | |style="text-align:right;"|פרק נ' מספר לשרש מעו' מצי‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|פרק נ"א מעו' לשרש מעו' ממס' | + | *chapter 51: <math>\scriptstyle ax^3=\sqrt[3]{c}</math> |
| + | |style="text-align:right;"|פרק נ"א מעו' לשרש מעו' ממס‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|פרק נ"ב מס' לשרש מעו' ממס' | + | *chapter 52: <math>\scriptstyle c={\color{red}{\sqrt[3]{ax^3}}}</math> |
| + | |style="text-align:right;"|פרק נ"ב מס' לשרש מעו' ממס‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|פרק נ"ג צינ' מצי' לשרש מעו' ממס' | + | *chapter 53: <math>\scriptstyle ax^4=\sqrt[3]{c}</math> |
| + | |style="text-align:right;"|פרק נ"ג צינ' מצי' לשרש מעו' ממס‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|פרק נ"ד מס' לשרש מעו' מצי' מצי' | + | *chapter 54: <math>\scriptstyle c=\sqrt[3]{ax^4}</math> |
| + | |style="text-align:right;"|פרק נ"ד מס' לשרש מעו' מצי' מצי‫' | ||
|- | |- | ||
| | | | ||
| + | *chapter 55: <math>\scriptstyle ax=\sqrt[3]{bx}</math> | ||
|style="text-align:right;"|פרק נ"ה דבר לשרש מעו' מדבר | |style="text-align:right;"|פרק נ"ה דבר לשרש מעו' מדבר | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|פרק נ"ו דבר לשרש מעו' מצי' | + | *chapter 56: <math>\scriptstyle bx=\sqrt[3]{ax^2}</math> |
| + | |style="text-align:right;"|פרק נ"ו דבר לשרש מעו' מצי‫' | ||
|- | |- | ||
| | | | ||
| + | *chapter 57: <math>\scriptstyle ax^3=\sqrt[3]{bx}</math> | ||
|style="text-align:right;"|פרק נ"ז מעו' לשרש מעו' מדבר | |style="text-align:right;"|פרק נ"ז מעו' לשרש מעו' מדבר | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|פרק נ"ח דב' לשרש מעו' מצי' מצי' | + | *chapter 58: <math>\scriptstyle bx=\sqrt[3]{ax^4}</math> |
| + | |style="text-align:right;"|פרק נ"ח דב' לשרש מעו' מצי' מצי‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|פרק נ"ט צי' לשרש מעו' מצי' | + | *chapter 59: <math>\scriptstyle ax^2=\sqrt[3]{bx^2}</math> |
| + | |style="text-align:right;"|פרק נ"ט צי' לשרש מעו' מצי‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|פרק ס' צי' לשרש מעו' ממעו' | + | *chapter 60: <math>\scriptstyle ax^2=\sqrt[3]{bx^3}</math> |
| + | |style="text-align:right;"|פרק ס' צי' לשרש מעו' ממעו‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|פרק ס"א צי' לשרש מעו' צי' מצי' | + | *chapter 61: <math>\scriptstyle ax^2=\sqrt[3]{bx^4}</math> |
| + | |style="text-align:right;"|פרק ס"א צי' לשרש מעו' צי' מצי‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|פרק ס"ב מעו' לשרש מעו' ממעו' | + | *chapter 62: <math>\scriptstyle ax^3=\sqrt[3]{bx^3}</math> |
| + | |style="text-align:right;"|פרק ס"ב מעו' לשרש מעו' ממעו‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|פרק ס"ג צי' מצי' לשרש מעו' ממעו' | + | *chapter 63: <math>\scriptstyle ax^4=\sqrt[3]{bx^3}</math> |
| + | |style="text-align:right;"|פרק ס"ג צי' מצי' לשרש מעו' ממעו‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|פרק ס"ד צי' מצי' לשרש מעו | + | *chapter 64: <math>\scriptstyle ax^4=\sqrt[3]{bx^4}</math> |
| + | |style="text-align:right;"|פרק ס"ד צי' מצי' לשרש מעו' צי' מצי‫' | ||
|- | |- | ||
| | | | ||
| + | *chapter 65: <math>\scriptstyle ax=c+\sqrt[3]{d}</math> | ||
|style="text-align:right;"|פרק ס"ה דבר למס' ולשרש מספר | |style="text-align:right;"|פרק ס"ה דבר למס' ולשרש מספר | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|פרק ס"ו צי' למס' ולשרש מעו' ממס' | + | *chapter 66: <math>\scriptstyle ax^2=c+\sqrt[3]{d}</math> |
| + | |style="text-align:right;"|פרק ס"ו צי' למס' ולשרש מעו' ממס‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|פרק ס"ז מעו' למס' ולש' מעו' ממס' | + | *chapter 67: <math>\scriptstyle ax^3=c+\sqrt[3]{d}</math> |
| + | |style="text-align:right;"|פרק ס"ז מעו' למס' ולש' מעו' ממס‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|פרק ס"ח צי' מצי' למס' ולש' מעו' ממס' | + | *chapter 68: <math>\scriptstyle ax^4=c+\sqrt[3]{d}</math> |
| + | |style="text-align:right;"|פרק ס"ח צי' מצי' למס' ולש' מעו' ממס‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|פרק ס"ט צי' מצי' ומעו' לצינ' | + | *chapter 69: <math>\scriptstyle ax^4+bx^3=cx^2</math> |
| + | |style="text-align:right;"|פרק ס"ט צי' מצי' ומעו' לצינ‫' | ||
|- | |- | ||
| | | | ||
| + | *chapter 70: <math>\scriptstyle ax^4+cx^2=bx^3</math> | ||
|style="text-align:right;"|פרק ע' צי' מצי' וצינ' למעוקב | |style="text-align:right;"|פרק ע' צי' מצי' וצינ' למעוקב | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|פרק ע"א צי' מצי' לצי' ולמעו' | + | *chapter 71: <math>\scriptstyle ax^4=cx^2+bx^3</math> |
| + | |style="text-align:right;"|פרק ע"א צי' מצי' לצי' ולמעו‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|פרק ע"ב צי' ודבר לשרש מספ' | + | *chapter 72: <math>\scriptstyle ax^2+bx=\sqrt{c}</math> |
| + | |style="text-align:right;"|פרק ע"ב צי' ודבר לשרש מספ‫' | ||
|- | |- | ||
| | | | ||
| + | *chapter 73: <math>\scriptstyle ax^2+\sqrt{c}=bx</math> | ||
|style="text-align:right;"|פרק ע"ג צינ' ושרש מס' לדבר | |style="text-align:right;"|פרק ע"ג צינ' ושרש מס' לדבר | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|פרק ע"ד דבר ושרש מספ' לצי' | + | *chapter 74: <math>\scriptstyle bx+\sqrt{c}=ax^2</math> |
| + | |style="text-align:right;"|פרק ע"ד דבר ושרש מספ' לצי‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|פרק ע"ה צי' מצי' וצי' לשרש מספ' | + | *chapter 75: <math>\scriptstyle ax^4+bx^2=\sqrt{c}</math> |
| + | |style="text-align:right;"|פרק ע"ה צי' מצי' וצי' לשרש מספ‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|פרק ע"ו צי' מצי' ושרש מס' לצי' | + | *chapter 76: <math>\scriptstyle ax^4+\sqrt{c}=bx^2</math> |
| + | |style="text-align:right;"|פרק ע"ו צי' מצי' ושרש מס' לצי‫' | ||
|- | |- | ||
| | | | ||
| + | *chapter 77: <math>\scriptstyle ax^4=bx^2+\sqrt{c}</math> | ||
|style="text-align:right;"|פרק ע"ז צי' מצי' לצי' וש' מספר | |style="text-align:right;"|פרק ע"ז צי' מצי' לצי' וש' מספר | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|פרק ע"ח דבר ושרש צי' למס' | + | *chapter 78: <math>\scriptstyle bx+\sqrt{ax^2}=c</math> |
| + | |style="text-align:right;"|פרק ע"ח דבר ושרש צי' למס‫' | ||
|- | |- | ||
| | | | ||
| + | *chapter 79: <math>\scriptstyle c+\sqrt{ax^2}=bx</math> | ||
|style="text-align:right;"|פרק ע"ט מס' ושרש צי' לדבר | |style="text-align:right;"|פרק ע"ט מס' ושרש צי' לדבר | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|פרק פ' דבר ומס' לשרש צינ' | + | *chapter 80: <math>\scriptstyle bx+c=\sqrt{ax^2}</math> |
| + | |style="text-align:right;"|פרק פ' דבר ומס' לשרש צינ‫' | ||
|- | |- | ||
| | | | ||
| + | *chapter 81: <math>\scriptstyle ax^2+\sqrt{bx^2}=cx</math> | ||
|style="text-align:right;"|פרק פ"א צי' ושרש צי' לדבר | |style="text-align:right;"|פרק פ"א צי' ושרש צי' לדבר | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|פרק פ"ב דבר ושרש צי' לצי' | + | *chapter 82: <math>\scriptstyle cx+\sqrt{bx^2}=ax^2</math> |
| + | |style="text-align:right;"|פרק פ"ב דבר ושרש צי' לצי‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|פרק פ"ג צי' ודבר לשרש צי' | + | *chapter 83: <math>\scriptstyle ax^2+cx=\sqrt{bx^2}</math> |
| + | |style="text-align:right;"|פרק פ"ג צי' ודבר לשרש צי‫' | ||
|- | |- | ||
| | | | ||
| + | *chapter 84: <math>\scriptstyle ax^2+\sqrt{bx^3}=cx</math> | ||
|style="text-align:right;"|פרק פ"ד צי' ושרש מעו' לדבר | |style="text-align:right;"|פרק פ"ד צי' ושרש מעו' לדבר | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|פרק פ"ה דבר ושרש מעו' לצי' | + | *chapter 85: <math>\scriptstyle cx+\sqrt{bx^3}=ax^2</math> |
| + | |style="text-align:right;"|פרק פ"ה דבר ושרש מעו' לצי‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|פרק פ"ו דבר וצי' לשרש מעו' | + | *chapter 86: <math>\scriptstyle cx+ax^2=\sqrt{bx^3}</math> |
| + | |style="text-align:right;"|פרק פ"ו דבר וצי' לשרש מעו‫' | ||
|- | |- | ||
| | | | ||
| + | *chapter 87: <math>\scriptstyle c+\sqrt{bx}=ax</math> | ||
|style="text-align:right;"|פרק פ"ז מס' ושרש דבר לדבר | |style="text-align:right;"|פרק פ"ז מס' ושרש דבר לדבר | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|פרק פ"ח מספ' ושרש צי' לצי' | + | *chapter 88: <math>\scriptstyle c+\sqrt{bx^2}=ax^2</math> |
| + | |style="text-align:right;"|פרק פ"ח מספ' ושרש צי' לצי‫' | ||
|- | |- | ||
| | | | ||
| + | *chapter 89: <math>\scriptstyle c+ax=\sqrt{bx}</math> | ||
|style="text-align:right;"|פרק פ"ט מספר ודבר לש' דבר | |style="text-align:right;"|פרק פ"ט מספר ודבר לש' דבר | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|פרק צ' מס' וצי' לשרש צינ' | + | *chapter 90: <math>\scriptstyle c+ax^2=\sqrt{bx^2}</math> |
| + | |style="text-align:right;"|פרק צ' מס' וצי' לשרש צינ‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|פרק צ"א מספ' ושרש מעו' למעו' | + | *chapter 91: <math>\scriptstyle c+\sqrt{bx^3}=ax^3</math> |
| + | |style="text-align:right;"|פרק צ"א מספ' ושרש מעו' למעו‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|פרק צ"ב מספ' ומעו' לשרש מעו' | + | *chapter 92: <math>\scriptstyle c+ax^3=\sqrt{bx^3}</math> |
| + | |style="text-align:right;"|פרק צ"ב מספ' ומעו' לשרש מעו‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|פרק צ"ג מס' וש' צי' מצי' לצי' | + | *chapter 93: <math>\scriptstyle c+\sqrt{bx^4}=ax^2</math> |
| + | |style="text-align:right;"|פרק צ"ג מס' וש' צי' מצי' לצי‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|פרק צ"ד מס' וצי' מצי' לשרש צי' מצי' | + | *chapter 94: <math>\scriptstyle c+ax^4=\sqrt{bx^4}</math> |
| + | |style="text-align:right;"|פרק צ"ד מס' וצי' מצי' לשרש צי' מצי‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|פרק צ"ה צי' ודבר לשרש מעו' ממס' | + | *chapter 95: <math>\scriptstyle ax^2+bx=\sqrt[3]{c}</math> |
| + | |style="text-align:right;"|פרק צ"ה צי' ודבר לשרש מעו' ממס‫' | ||
|- | |- | ||
| | | | ||
| + | *chapter 96: <math>\scriptstyle ax^2+\sqrt[3]{c}=bx</math> | ||
|style="text-align:right;"|פרק צ"ו צי' וש' מעו' ממס' לדבר | |style="text-align:right;"|פרק צ"ו צי' וש' מעו' ממס' לדבר | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|פרק צ"ז דבר וש' מעו' ממס' לצי' | + | *chapter 97: <math>\scriptstyle bx+\sqrt[3]{c}=ax^2</math> |
| + | |style="text-align:right;"|פרק צ"ז דבר וש' מעו' ממס' לצי‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|פרק צ"ח צי' מצי' וצי' לש' מעו' ממס' | + | *chapter 98: <math>\scriptstyle ax^4+bx^2=\sqrt[3]{c}</math> |
| + | |style="text-align:right;"|פרק צ"ח צי' מצי' וצי' לש' מעו' ממס‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|פרק צ"ט צי' מצי' וש' מעו' ממס' לצי' | + | *chapter 99: <math>\scriptstyle ax^4+\sqrt[3]{c}=bx^2</math> |
| + | |style="text-align:right;"|פרק צ"ט צי' מצי' וש' מעו' ממס' לצי‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|פרק ק' צי' מצי' לצי' ולש' מעו' ממס' | + | *chapter 100: <math>\scriptstyle ax^4=bx^2+\sqrt[3]{c}</math> |
| − | + | |style="text-align:right;"|פרק ק' צי' מצי' לצי' ולש' מעו' ממס‫' | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|פרק | + | *chapter 101: <math>\scriptstyle ax+b=\sqrt{c}</math> |
| + | |style="text-align:right;"|פרק ק"א דבר ומס' לש' מספר | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|פרק | + | *chapter 102: <math>\scriptstyle ax+\sqrt{c}=b</math> |
| + | |style="text-align:right;"|פרק ק"ב דבר ושרש מספ' למס‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|פרק | + | *chapter 103: <math>\scriptstyle ax^2+b=\sqrt{c}</math> |
| + | |style="text-align:right;"|פרק ק"ג צי' ומס' לשרש מספ‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|פרק | + | *chapter 104: <math>\scriptstyle ax^2+\sqrt{c}=b</math> |
| + | |style="text-align:right;"|פרק ק"ד צי' ושרש מס' למספר | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|פרק | + | *chapter 105: <math>\scriptstyle ax^3+b=\sqrt{c}</math> |
| + | |style="text-align:right;"|פרק ק"ה מעו' ומס' לש' מספר | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|פרק ק" | + | *chapter 106: <math>\scriptstyle ax^3+\sqrt{c}=b</math> |
| + | |style="text-align:right;"|פרק ק"ו מעו' ושרש מס' למס‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|פרק | + | *chapter 107: <math>\scriptstyle ax^4+b=\sqrt{c}</math> |
| + | |style="text-align:right;"|‫<ref>195r</ref>פרק ק"ז צי' מצי' ומס' לש' מספר | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|פרק | + | *chapter 108: <math>\scriptstyle ax^4+\sqrt{c}=b</math> |
| + | |style="text-align:right;"|פרק ק"ח צי' מצי' ושרש מס' למס‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|פרק | + | *chapter 109: <math>\scriptstyle ax+\sqrt{bx}=\sqrt{c}</math> |
| + | |style="text-align:right;"|פרק ק"ט דבר וש' דבר לש' מספר | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|פרק | + | *chapter 110: <math>\scriptstyle ax+\sqrt{c}=\sqrt{bx}</math> |
| + | |style="text-align:right;"|פרק קי' דבר וש' מס' לש' דבר | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 111: <math>\scriptstyle\sqrt{c}+\sqrt{bx}=ax</math> |
| + | |style="text-align:right;"|פרק קי"א שרש מס' וש' דבר לדבר‫' | ||
|- | |- | ||
| | | | ||
| − | + | *chapter 112: <math>\scriptstyle ax^2+\sqrt{bx^2}=\sqrt{c}</math> | |
| − | + | |style="text-align:right;"|פרק קי"ב צי' וש' צי' לשרש מספר | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | { | ||
| − | |||
| − | |style="text-align:right;"| | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 113: <math>\scriptstyle ax^2+\sqrt{c}=\sqrt{bx^2}</math> |
| + | |style="text-align:right;"|פרק קי"ג צי' וש' מס' לשרש צינ‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 114: <math>\scriptstyle\sqrt{bx^2}+\sqrt{c}=ax^2</math> |
| − | + | |style="text-align:right;"|פרק קי"ד שרש צי' וש' מספ' לצי‫' | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 115: <math>\scriptstyle ax^3+\sqrt{bx^3}=\sqrt{c}</math> |
| + | |style="text-align:right;"|פרק קט"ו מעו' וש' מעו' לש' מס‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 116: <math>\scriptstyle ax^3+\sqrt{c}=\sqrt{bx^3}</math> |
| + | |style="text-align:right;"|פרק קי"ו מעו' וש' מספ' לש' מעו‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 117: <math>\scriptstyle\sqrt{bx^3}+\sqrt{c}=ax^3</math> |
| − | + | |style="text-align:right;"|פרק קי"ז שרש מעו' ושרש מס' למעו‫' | |
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 118: <math>\scriptstyle ax^4+\sqrt{bx^4}=\sqrt{c}</math> |
| + | |style="text-align:right;"|פרק קי"ח צי' מצי' וש' צי' מצי' לש' מס‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 119: <math>\scriptstyle ax^4+\sqrt{c}=\sqrt{bx^4}</math> |
| + | |style="text-align:right;"|פרק קי"ט צי' מצי' וש' מס' לש' צי' מצי‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 120: <math>\scriptstyle\sqrt{bx^4}+\sqrt{c}=ax^4</math> |
| − | + | |style="text-align:right;"|פרק ק"כ ש' צי' מצי' וש' מס' לצי' מצי‫' | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 121: <math>\scriptstyle ax+\sqrt{bx}=\sqrt[3]{c}</math> |
| + | |style="text-align:right;"|פרק קכ"א דבר וש' דבר לש' מעו' ממס‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 122: <math>\scriptstyle ax+\sqrt[3]{c}=\sqrt{bx}</math> |
| + | |style="text-align:right;"|פרק קכ"ב דבר וש' מעו' ממס' לש' דב‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 123: <math>\scriptstyle\sqrt{ax^2}+\sqrt[3]{c}=bx</math> |
| − | + | |style="text-align:right;"|פרק קכ"ג ש' צי' וש' מעו' ממס' לדבר | |
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 124: <math>\scriptstyle ax^2+\sqrt{bx^2}=\sqrt[3]{c}</math> |
| + | |style="text-align:right;"|פרק קכ"ד צי' וש' צי' לש' מעו' ממס‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 125: <math>\scriptstyle ax^2+\sqrt[3]{c}=\sqrt{bx^2}</math> |
| + | |style="text-align:right;"|פרק קכ"ה צי' וש' מעו' ממס' לש' צי‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 126: <math>\scriptstyle\sqrt{bx^2}+\sqrt[3]{c}=ax^2</math> |
| − | + | |style="text-align:right;"|פרק קכ"ו ש' צי' וש' מעו' ממס' לצי‫' | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 127: <math>\scriptstyle ax^3+\sqrt{bx^3}=\sqrt[3]{c}</math> |
| + | |style="text-align:right;"|פרק קכ"ז מעו' וש' מעו' לש' מעו' ממס‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 128: <math>\scriptstyle ax^3+\sqrt[3]{c}={\color{red}{\sqrt{bx^3}}}</math> |
| − | + | |style="text-align:right;"|פרק קכ"ח מעו' וש' מעו' ממס' למעו‫' | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 129: <math>\scriptstyle\sqrt{bx^3}+\sqrt[3]{c}=ax^3</math> |
| + | |style="text-align:right;"|פרק קכ"ט ש' מעו' וש' מעו' ממס' למעו‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 130: <math>\scriptstyle ax^4+\sqrt{bx^4}=\sqrt[3]{c}</math> |
| + | |style="text-align:right;"|פרק ק"ל צי' מצי' וש' צי' מצי' לש' מעו' מס‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 131: <math>\scriptstyle ax^4+\sqrt[3]{c}=\sqrt{bx^4}</math> |
| − | + | |style="text-align:right;"|פרק קל"א צי' מצי' וש' מעו' מס' לש' צי' מצי‫' | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 132: <math>\scriptstyle\sqrt{bx^4}+\sqrt[3]{c}=ax^4</math> |
| + | |style="text-align:right;"|פרק קל"ב ש' צי' מצי' וש' מעו' מס' לצי' מצי‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 133: <math>\scriptstyle bx^2+cx=\sqrt{ax^4}=</math> |
| + | |style="text-align:right;"|פרק קל"ג צי' ודבר לש' צי' מצינסו | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 134: <math>\scriptstyle{\color{red}{bx^2}}+\sqrt{ax^4}=cx</math> |
| − | + | |style="text-align:right;"|פרק קל"ד דבר וש' מצי' דצי' לדבר | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 135: <math>\scriptstyle cx+\sqrt{ax^4}=bx^2</math> |
| + | |style="text-align:right;"|פרק קל"ה דבר וש' צי' מצי' לצי‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 136: <math>\scriptstyle bx+d=\sqrt[3]{c}</math> |
| + | |style="text-align:right;"|פרק קל"ו דבר ומס' לש' מעו' מס‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 137: <math>\scriptstyle bx+\sqrt[3]{c}=d</math> |
| − | + | |style="text-align:right;"|פרק קל"ז דבר וש' מעו' מס' למס‫' | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 138: <math>\scriptstyle {\color{red}{ax^2}}+d=\sqrt[3]{c}</math> |
| + | |style="text-align:right;"|פרק קל"ח דבר ומס' לש' מעו' מס‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 139: <math>\scriptstyle ax^2+\sqrt[3]{c}=d</math> |
| + | |style="text-align:right;"|פרק קל"ט צי' וש' מעו' מס' למס‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 140: <math>\scriptstyle ax^3+d=\sqrt[3]{c}</math> |
| − | + | |style="text-align:right;"|פרק ק"מ מעו' ומס' לש' מעו' מס‫' | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | ק | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 141: <math>\scriptstyle ax^3+\sqrt[3]{c}=d</math> |
| + | |style="text-align:right;"|פרק קמ"א מעו' וש' מעו' מס' למס‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 142: <math>\scriptstyle ax^4+d=\sqrt[3]{c}</math> |
| + | |style="text-align:right;"|פרק קמ"ב צי' דצי' ומס' לש' מעו' מס‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 143: <math>\scriptstyle ax^4+\sqrt[3]{c}=d</math> |
| − | + | |style="text-align:right;"|פרק קמ"ג צי' מצי' וש' מעו' מס' למס‫' | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 144: <math>\scriptstyle\sqrt{ax^2}+bx=\sqrt{c}</math> |
| + | |style="text-align:right;"|פרק קמ"ד ש' מצי' ודבר לש' מספר | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 145: <math>\scriptstyle\sqrt{ax^2}+\sqrt{c}=bx</math> |
| + | |style="text-align:right;"|פרק קמ"ה ש' צי' וש' מספ' לדבר | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 146: <math>\scriptstyle bx+\sqrt{c}=\sqrt{ax^2}</math> |
| − | + | |style="text-align:right;"|פרק קמ"ו דבר וש' מס' לש' צי‫' | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 147: <math>\scriptstyle\sqrt{ax^2}+bx=\sqrt[3]{c}</math> |
| + | |style="text-align:right;"|פרק קמ"ז ש' צי' ודבר לש' מעו' מס‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 148: <math>\scriptstyle\sqrt{ax^2}+\sqrt[3]{c}=bx</math> |
| + | |style="text-align:right;"|פרק קמ"ח ש' צי' וש' מעו' מס' לדבר | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 149: <math>\scriptstyle bx+\sqrt[3]{c}=\sqrt{ax^2}</math> |
| − | + | |style="text-align:right;"|פרק קמ"ט דבר וש' מעו' מס' לש' צי‫' | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 150: <math>\scriptstyle bx^2+\sqrt{ax^4}=c</math> |
| + | |style="text-align:right;"|פרק ק"ן צי' וש' צי' מצי' למספר | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 151: <math>\scriptstyle c+\sqrt{ax^4}=bx^2</math> |
| + | |style="text-align:right;"|פרק קנ"א מס' וש' צינ' מצי' לצינסו | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 152: <math>\scriptstyle bx^2+c=\sqrt{ax^4}</math> |
| − | + | |style="text-align:right;"|פרק קנ"ב צי' ומס' לש' צי' מצי‫' | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 153: <math>\scriptstyle bx^2+\sqrt{ax^4}=\sqrt{c}</math> |
| + | |style="text-align:right;"|פרק קנ"ג צי' וש' צי' מצי' לש' מספ‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 154: <math>\scriptstyle\sqrt{ax^4}+\sqrt{c}=bx^2</math> |
| + | |style="text-align:right;"|פרק קנ"ד ש' צי' מצי' וש' מס' לצי‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 155: <math>\scriptstyle bx^2+\sqrt{c}=\sqrt{ax^4}</math> |
| − | + | |style="text-align:right;"|פרק קנ"ה צי' וש' מס' לש' צי' מצי‫' | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 156: <math>\scriptstyle bx^2+\sqrt{ax^4}=\sqrt[3]{c}</math> |
| + | |style="text-align:right;"|פרק קנ"ו צי' וש' צי' מצי' לש' מעו' מס‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 157: <math>\scriptstyle\sqrt{ax^4}+\sqrt[3]{c}=bx^2</math> |
| + | |style="text-align:right;"|פרק קנ"ז ש' צי' מצי' וש' מעו' מס' לצי‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 158: <math>\scriptstyle ax^2+\sqrt[3]{c}=\sqrt{bx^4}</math> |
| − | + | |style="text-align:right;"|פרק קנ"ח צי' וש' מעו' מס' לש' צי' מצי‫' | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 159: <math>\scriptstyle\sqrt[3]{bx}=c</math> |
| + | |style="text-align:right;"|פרק קנ"ט ש' מעו' דבר למספר | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 160: <math>\scriptstyle\sqrt[3]{ax^2}=c</math> |
| + | |style="text-align:right;"|פרק ק"ס ש' מעו' צי' למס‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 161: <math>\scriptstyle\sqrt[3]{ax^3}=c</math> |
| − | + | |style="text-align:right;"|פרק קס"א ש' מעו' מעו' למספר | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 162: <math>\scriptstyle\sqrt[3]{ax^4}=c</math> |
| + | |style="text-align:right;"|פרק קס"ב ש' מעו' צי' מצי' למס‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 163: <math>\scriptstyle\sqrt[3]{bx}=\sqrt{c}</math> |
| + | |style="text-align:right;"|פרק קס"ג ש' מעו' דבר לש' מס‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 164: <math>\scriptstyle\sqrt[3]{ax^2}=\sqrt{c}</math> |
| − | + | |style="text-align:right;"|פרק קס"ד ש' מעו' צי' לש' מספ‫' | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 165: <math>\scriptstyle\sqrt[3]{ax^3}=\sqrt{c}</math> |
| + | |style="text-align:right;"|פרק קס"ה ש' מעו' ממעו' לש' מס‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 166: <math>\scriptstyle\sqrt[3]{ax^4}=\sqrt{c}</math> |
| + | |style="text-align:right;"|פרק קס"ו ש' מעו' צי' מצי' לש' מס‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 167: <math>\scriptstyle\sqrt{bx}=\sqrt[3]{c}</math> |
| − | + | |style="text-align:right;"|‫<ref>Paris 195v</ref>פרק קס"ז שרש דבר לש' מעו' מס‫' | |
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 168: <math>\scriptstyle\sqrt{ax^2}=\sqrt[3]{c}</math> |
| + | |style="text-align:right;"|פרק קס"ח שרש צי' לש' מעו' מספ‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 169: <math>\scriptstyle\sqrt{ax^3}=\sqrt[3]{c}</math> |
| + | |style="text-align:right;"|פרק קס"ט שרש מעו' לש' מעו' מס‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 170: <math>\scriptstyle\sqrt{ax^4}=\sqrt[3]{c}</math> |
| − | + | |style="text-align:right;"|פרק ק"ע ש' צי' מצי' לש' מעו' מספ‫' | |
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 171: <math>\scriptstyle\sqrt{ax}=\sqrt[3]{bx}</math> |
| + | |style="text-align:right;"|פרק קע"א ש' דבר לש' מעו' דבר | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 172: <math>\scriptstyle\sqrt{ax^2}=\sqrt[3]{bx^2}</math> |
| + | |style="text-align:right;"|פרק קע"ב ש' צי' לש' מעו' צינסו | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 173: <math>\scriptstyle\sqrt{ax^3}=\sqrt[3]{bx^3}</math> |
| − | + | |style="text-align:right;"|פרק קע"ג ש' מעו' לש' מעו' ממעו‫' | |
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 174: <math>\scriptstyle\sqrt{ax^4}=\sqrt[3]{bx^4}</math> |
| + | |style="text-align:right;"|פרק קע"ד ש' צי' מצי' לש' מעו' צי' מצי‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 175: <math>\scriptstyle\sqrt{bx}=\sqrt[3]{ax^2}</math> |
| + | |style="text-align:right;"|פרק קע"ה ש' דבר לש' מעו' צי‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 176: <math>\scriptstyle\sqrt{bx}=\sqrt[3]{ax^3}</math> |
| − | + | |style="text-align:right;"|פרק קע"ו שרש דבר לש' מעו' המעו‫' | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 177: <math>\scriptstyle\sqrt{ax}=\sqrt[3]{bx^4}</math> |
| + | |style="text-align:right;"|פרק קע"ז ש' דבר לש' מעו' צי' מצי‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 178: <math>\scriptstyle\sqrt{ax^2}=\sqrt[3]{bx}</math> |
| + | |style="text-align:right;"|פרק קע"ח ש' צי' לש' מעו' מדבר | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 179: <math>\scriptstyle\sqrt{ax^3}=\sqrt[3]{bx}</math> |
| − | + | |style="text-align:right;"|פרק קע"ט ש' מעו' לש' מעו' מדבר | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 180: <math>\scriptstyle\sqrt{ax^4}=\sqrt[3]{bx^2}</math> |
| + | |style="text-align:right;"|פרק ק"פ ש' צי' מצי' לש' מעו' מצי‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 181: <math>\scriptstyle\sqrt{ax^3}=\sqrt[3]{bx^4}</math> |
| + | |style="text-align:right;"|פרק קפ"א ש' מעו' לש' מעו' צי' מצי‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 182: <math>\scriptstyle\sqrt{ax^4}=\sqrt[3]{bx^3}</math> |
| − | + | |style="text-align:right;"|פרק קפ"ב ש' צי' מצי' לש' מעו' המעו‫' | |
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 183: <math>\scriptstyle{\color{red}{ax^2+bx}}=c+\sqrt{d}</math> |
| + | |style="text-align:right;"|פרק קפ"ג צי' מצי' למס' ולש' מס‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 184: <math>\scriptstyle ax^2+c+\sqrt{d}=bx</math> |
| + | |style="text-align:right;"|פרק קפ"ד צי' ומס' וש' מס' לדבר | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 185: <math>\scriptstyle bx+c+\sqrt{d}=ax^2</math> |
| − | + | |style="text-align:right;"|פרק קפ"ה דבר ומס' וש' מס' לצי‫' | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 186: <math>\scriptstyle ax^2+bx=c+\sqrt[3]{d}</math> |
| + | |style="text-align:right;"|פרק קפ"ו צי' ודבר למס' ולש' מעו' מס‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 187: <math>\scriptstyle ax^2+c+\sqrt[3]{d}=bx</math> |
| + | |style="text-align:right;"|פרק קפ"ז צי' ומס' וש' מעו' מס' לדבר | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 188: <math>\scriptstyle bx+c+\sqrt[3]{d}=ax^2</math> |
| − | + | |style="text-align:right;"|פרק קפ"ח דבר ומס' וש' מעו' מס' לצי‫' | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 189: <math>\scriptstyle ax^4+bx^2=c+\sqrt{d}</math> |
| + | |style="text-align:right;"|פרק קפ"ט צי' מצי' וצי' למס' וש' מס‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 190: <math>\scriptstyle ax^4+c+\sqrt{d}=bx^2</math> |
| + | |style="text-align:right;"|פרק ק"ץ צי' מצי' ומס' וש' מס' לצי‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 191: <math>\scriptstyle bx^2+c+\sqrt{d}=ax^4</math> |
| − | + | |style="text-align:right;"|פרק קצ"א צי' ומס' וש' מס' לצי' מצי‫' | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 192: <math>\scriptstyle ax^4+bx^2=c+\sqrt[3]{d}</math> |
| + | |style="text-align:right;"|פרק קצ"ב צי' מצי' וצי' למס' וש' מעו' ממס‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 193: <math>\scriptstyle ax^4+c+\sqrt[3]{d}=bx^2</math> |
| − | + | |style="text-align:right;"|פרק קצ"ג צי' מצי' ומס' וש' מעו' מס' לצי‫' | |
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *chapter 194: <math>\scriptstyle bx^2+c+\sqrt[3]{d}=ax^4</math> |
| + | |style="text-align:right;"|פרק קצ"ד צי' ומס' וש' מעו' מס' לצי' מצי‫' | ||
|- | |- | ||
| − | | | + | |The chapters mentioned above are laid out in general rules, as seen further in the booklet, on page 121. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<big>הפרקים</big> הנזכרים למעלה הם סודרו מ{{#annot:term|222,1401|Z8ME}}סדרים{{#annotend:Z8ME}} כוללים כנראה בהמשך הקנטריס בעלה קכ"א |
|- | |- | ||
| − | | | + | |Still further, a few chapters are laid out in non inclusive rules, while these rules are truthful for the rules that are given for the amendments of the aforementioned chapters, and these are: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<big>עוד</big> אחר זה אמשיך כמה פרקים אשר הם מסודרים מסדרים בלתי כוללים עם שהסדרים ההם הם אמתיים ל{{#annot:term|222|N1Rc}}משפטים{{#annotend:N1Rc}} המגיעים לתיקוניהם של הפרקים הנזכרים והם אלו אשר אכתוב |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *Chapter 1: <math>\scriptstyle cx+bx^2+ax^3=n</math> |
| + | |style="text-align:right;"|פרק א' דבר וצי' ומעו' למספ‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *Chapter 2: <math>\scriptstyle dx+cx^2+bx^3+ax^4=n</math> |
| − | + | |style="text-align:right;"|פרק ב' דבר וצי' ומעו' וצי' מצי' למס‫' | |
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *Chapter 3: <math>\scriptstyle cx+ax^4=n+bx^3</math> |
| − | + | |style="text-align:right;"|פרק ג' דב' וצי' מצי' למס' ומעו‫' | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *Chapter 4: <math>\scriptstyle dx+ax^4=n+cx^2+bx^3</math> |
| + | |style="text-align:right;"|פרק ד' דבר וצי מצי' למס' ולצי' ולמעו‫' | ||
|- | |- | ||
| − | | | + | |Then, after these chapters, the rules for extracting square root and cube root of any number are given: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<big>עוד</big> ימשכו אחר אלו הפרקים {{#annot:term|222,1401|i8RG}}סדר{{#annotend:i8RG}} הוצאת ש' מרובע ושרש מעוקב מכל מספר שיהיה |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *Chapter 1: rule for extracting the square root |
| + | |style="text-align:right;"|פרק א' סדר למצא שרש המרובע | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *Chapter 2: rule for extracting the cube root |
| − | + | |style="text-align:right;"|פרק ב' הסדר למצא שרש המעוקב | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | שרש | ||
|- | |- | ||
| − | | | + | |Know that after the above mentioned rules, many other calculations are calculated, which are beautiful and subtle. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<big>ודע</big> כי אחרי הסדרים הנזכרים {{#annot:term|229,1269|fUWL}}יחשבו{{#annotend:fUWL}} {{#annot:term|228,1200|09KJ}}חשבונות{{#annotend:09KJ}} אחרים רבים שהם יפים ודקים |
|- | |- | ||
| − | | | + | |Some of them can be defined with the aforesaid rules, |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אבל קצתם יתכן לשומם עם הסדרים הנזכרים |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |and some of them cannot, yet they are nice and delightful. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וקצתם לא יתכן אבל הם יפים ומענגים |
| + | |} | ||
| + | {| | ||
|- | |- | ||
| | | | ||
| − | + | ||
| − | + | == Prologue == | |
| − | + | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| | | | ||
| − | |||
|- | |- | ||
| − | | | + | |In the name of the Lord, Amen |
| − | |style="text-align:right;"| | + | |style="width:45%;text-align:right;"|‫<ref>1r</ref><big>בשם</big> השם אמן |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |This book was translated from another book, first written on November 9, 1344 (Christian Calendar). |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|זה הספר נעתק מספר אחר אשר נכתב ראשונה בט' נובימ' אלף שמ"ד לחשבו' הנצרים |
|- | |- | ||
| − | | | + | |Then, Jacomo di Ierushali da Litovilana, who lives in Mantua on the road of Unicorno, close to the Church of San Barnaba, began writing it on Saturday, May 3, 1429. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואחר זה התחילו לכתוב אותו יקומו דירושילי דליטובילאנה הדר בעיר מנטואה במסלת האוניקורנו קרוב לקדש ס' בירנבי ביום שבת ג' מאיו [אלף תכ"ט]‫<ref>om.</ref> |
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |In which many rules beneficial in calculations are kept, as we can see further in this book. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אשר בו יוחזקו סדרים רבים בעלי תועלת מחשבונות כאשר נוכל לראות בהמשך זה הספר |
|- | |- | ||
| − | | | + | |I, Mordecai Finzi, started translating it here, in Mantua, from the Christian language to Hebrew, for the benefit of our people, on Wednesday, November 24, year 5234 from the creation [= 1473 C.E.]. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואני מרדכי פינצי התחלתי להעתיקו הנה מנטואה מן הנצרי אל העברי לתועלת בני עמנו ביום רביעי כ"ד בנובימ' שנת ה' אלפי' ורל"ד ליצירה |
| − | |||
| − | |||
| − | ד' | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | ד | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |I have trusted the Lord, I shall not stumble. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ובה' בטחתי לא אמעד‫<ref>תהילים כו, א</ref> |
| + | |} | ||
| + | {| | ||
|- | |- | ||
| | | | ||
| − | + | ||
| − | + | == Roots == | |
| + | |||
| | | | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |Hereinafter, I start the necessary rules of multiplication and division of roots, as well as summing roots together, subtracting a root from another root, extracting expressible roots of square and cubic numbers, and many other useful rules by which the calculations of the craftsmen are known. |
| − | |style="text-align:right;"| | + | |style="width:45%; text-align:right;"|<big>בכאן</big> אתחיל הסדרי' הראויים ב{{#annot:term|156,1256|UTLc}}הכאה{{#annotend:UTLc}} וב{{#annot:term|157,1221|fhJq}}חלוקה{{#annotend:fhJq}} לשרשים וג"כ לחבר שרשי' יחד ולהוציא שרש משרש אחר וג"כ למצוא שרשי מספרי מרובע ומעוקב {{#annot:term|1075,2192|tf2L}}המדוברים{{#annotend:tf2L}} וסדרי' אחרי' רבים דקים ומועילים אשר עמהם יוכרו חשבונו' בעלי אומנות |
|- | |- | ||
| − | | | + | |First, we start with understanding what are square and cube roots of a number, why they are called roots, I mean what is the essence of the root of a number, whether a square or a cube root, and in what way the roots yield a number. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<big>וראשונה</big> נתחיל להבין מה הוא השרש המרובע וג"כ שרש מעוקב ממספר ולמה נקראים שרשים רצוני מהו <sup>{{#annot:term|1058|pUfD}}עצם{{#annotend:pUfD}}</sup> שרש המספר בין שיהיה {{#annot:term|559,1263|JaBu}}שרש מרובע{{#annotend:JaBu}} או {{#annot:term|558,1828|7PFn}}שרש מעוקב{{#annotend:7PFn}} ובאיזה אופן השרשי' יעשו מספר |
| − | |||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |One should first know the nature of the root - as every number is multiplied by itself, the square root is the product of its multiplication. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<big>וראשונה</big> ראוי לדעת טבע השרש בהיות כי כל מספר מוכה בעצמו הנה הוא שרש מרובע {{#annot:term|241,1241|dSq6}}העולה מהכאתו{{#annotend:dSq6}} |
|- | |- | ||
| − | | | + | |It should be known that every number has a square and cube root either visible or hidden. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וראוי לדעת כי לכל מספר יש לו שרש מרובע <s>או</s> ומעוקב גלוי או נעלם |
|- | |- | ||
| − | | | + | |But, not every number has a visible square and cube root. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אבל אין לכל מספר שרש <s>גלוי</s> מרובע גלוי גם לא מעוקב |
| − | |||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |The numbers that do not have a visible square nor cube root are called deaf or mute, whether square or cube. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אבל אותם המספרי' אשר לא נמצא להם שרש גלוי <sup>לא</sup> מרובע ולא מעוקב נקרא חרש או אלם מרובע יהיה או מעוקב |
|- | |- | ||
| − | | | + | |Since they cannot be expressed or heard by a visible number. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|מפני כי {{#annot:term|2370,2193|TruA}}לא ידובר בו{{#annotend:TruA}} ולא יאוזן במספר גלוי |
|- | |- | ||
| − | | | + | |They are also called continuous or concealed. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וג"כ יקראו תמידי' או לא גלויים |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |Since the numbers are formed by them, but their expression is hidden. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|מפני כי בהם יקוימו המספרי' ונעלם הבטוי בו |
|- | |- | ||
| − | | | + | |Know that every number that is multiplied by itself, then the product is multiplied by that same number, which is its root, is a cube. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<big>ודע</big> כי כל מספר שיוכה בעצמו והעולה יוכה במספר בעצמו שהם שרשיו הוא מעוקב |
|- | |- | ||
| − | | | + | |Since we have told you the nature of the square root, then of the cube root, and the way each of them by itself is found from the number, for which they are called roots, I wish to give you examples for square roots: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ובהיות שאמרנו לך למעלה טבע שרש המרובע ואחר ג"כ משרש המעוקב ובאיזה אופן כל אחד לבדו יגיע המספר אשר בעד המספר ההוא יקראו שרשים רציתי {{#annot:term|197,1712|kKU3}}לשים לך דמיון{{#annotend:kKU3}} השרש מרובע |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *One is a root of itself, because, when it is multiplied by itself the result is no other than itself. |
| + | :<math>\scriptstyle{\color{blue}{1^2=1\longrightarrow1=\sqrt{1}}}</math> | ||
| + | :<math>\scriptstyle{\color{blue}{}}</math> | ||
| + | |style="text-align:right;"|האחד הוא שרש לעצמו מפני כי כשיוכה בעצמו לא יגיע רק עצמו רצוני אחד | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *Two is a square root of four, and three of nine. Because when 2 is multiplied by itself, the result is four, and 3 by itself yields nine. |
| + | :<math>\scriptstyle{\color{blue}{2^2=4\longrightarrow2=\sqrt{4}}}</math> | ||
| + | :<math>\scriptstyle{\color{blue}{3^2=9\longrightarrow3=\sqrt{9}}}</math> | ||
| + | |style="text-align:right;"|ושנים הוא שרש מרובע מארבעה ושלשה מתשעה מפני כי כשיוכה ב' בעצמו יעלה ארבעה וג' בעצמו יעלה תשעה | ||
|- | |- | ||
| − | | | + | |In this manner: any root or number is a root of its product by itself |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ובזה האופן כל שרש או מספר הוא שרש מאשר יעלה הכאתו בעצמו |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |Examples for cube roots: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<big>ו{{#annot:term|197,1712|Vk8y}}דמיון{{#annotend:Vk8y}}</big> שרש מעוקב |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *One is a cube root of itself, because when it is multiplied by itself, then the product, which is one, is multiplied again by one, the result is no other than itself, meaning one. |
| − | + | :<math>\scriptstyle{\color{blue}{1^3=1^2\sdot1=1\sdot1=1\longrightarrow1=\sqrt[3]{1}}}</math> | |
| − | + | |style="text-align:right;"|האחד הוא שרש מעוקב גם כן מעצמו ר"ל מאחד<br> | |
| − | + | מפני כי כשיוכה אחד על עצמו ואחר זה {{#annot:term|876,1241|JBFu}}העולה{{#annotend:JBFu}} שהוא אחד יוכה ג"כ במספר ההוא לא יעלה רק עצמו רצוני אחד | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *Two is a cube root of eight, and three of 27. Because when 2 is multiplied by itself, the result is 4, and this product, meaning 4, multiplied by the said number, which is two, is eight. When three is multiplied by itself, the result is nine, and the nine multiplied by the said number, which is three, is 27. |
| + | :<math>\scriptstyle{\color{blue}{2^2\sdot2=4\sdot2=8\longrightarrow2=\sqrt[3]{8}}}</math> | ||
| + | :<math>\scriptstyle{\color{blue}{3^2\sdot3=9\sdot3=27\longrightarrow3=\sqrt[3]{27}}}</math> | ||
| + | |style="text-align:right;"|ושנים הוא שרש מעוקב לשמנה ושלשה לכ"ז מפני כי כשיוכה ב' בעצמו יעלה ד' ‫<ref>1v</ref>וזה העולה רצוני ד' יוכה במספר האמור הוא שנים יעלה שמנה וכשיוכה שלשה על עצמו יעלה תשעה וזה התשעה יוכה במספר האמור הוא שלשה יעלה כ"ז | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *Therefore, one is a square root of one and a cube root of one. |
| − | + | :<math>\scriptstyle{\color{blue}{1=\sqrt{1}=\sqrt[3]{1}}}</math> | |
| − | + | |style="text-align:right;"|ולזה האחד הוא שרש מרובע לאחד ושרש מעוקב לאחד | |
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *Two is a square root of four and a cube root of eight. |
| + | :<math>\scriptstyle{\color{blue}{2=\sqrt{4}=\sqrt[3]{8}}}</math> | ||
| + | |style="text-align:right;"|ושנים הוא שרש מרובע לארבעה ושרש מעוקב לשמנה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *3 is a square root of nine and a cube root of 27. |
| + | :<math>\scriptstyle{\color{blue}{3=\sqrt{9}=\sqrt[3]{27}}}</math> | ||
| + | |style="text-align:right;"|וג' הוא שרש מרובע לתשעה ושרש מעוקב לכ"ז | ||
|- | |- | ||
| − | | | + | |In this manner the square numbers and the cube numbers are found, and their roots are understood. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ובזה האופן ימצאו {{#annot:term|86,1263|o04g}}המספרי' המרובעי'{{#annotend:o04g}} ו{{#annot:term|91,1828|4G8I}}המספרי' המעוקבי'{{#annotend:4G8I}} והבנת שרשיהם |
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |In this order endlessly. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ועל זה הסדר עד לאין תכלית |
| + | |} | ||
| + | {| | ||
|- | |- | ||
| | | | ||
| − | + | === <span style=color:Green>Multiplication of Roots</span> === | |
| − | + | ||
| | | | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |After you have seen the nature of the roots and the essence of square and cube numbers, and the extraction of their roots, |
| − | |style="text-align:right;"| | + | |style="width:45%;text-align:right;"|<big>אחר</big> אשר ראית טבע השרשי' ו{{#annot:term|1058|FyrU}}עצמות{{#annotend:FyrU}} המספרי' המרובעי' והמספרי' המעוקבי' איך יצאו משרשיהם |
|- | |- | ||
| − | | | + | |From here on, I wish to show you how to multiply one root by another root, or a number by a root, or a number and a root by a number and a root, or a number minus a root by a number minus a root, and any other manner that can be carried out, as you will see in the following teaching of the aforementioned multiplication. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|מכאן ולהבא רצוני להראותך כיצד תכפול שרש אחד בשרש אחר או מספר בשרש או מספר ושרש במספר ושרש או מספר {{#annot:term|879,1366|wS2q}}פחות{{#annotend:wS2q}} שרש במספר פחות שרש וגם כן בכל אופן אחר אשר יוכל להגיע כפי אשר תראה בלמוד הבא בכפל הנזכר |
| + | |} | ||
| + | {| | ||
|- | |- | ||
| | | | ||
| − | + | ==== <span style=color:Green>Multiplication of a Root by a Root</span> ==== | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| | | | ||
| − | |||
|- | |- | ||
| − | | | + | |First I want to show you how we multiply one root simply by another: |
| − | |style="text-align:right;"| | + | |style="width:45%;text-align:right;"|<big>וראשונה</big> רצוני להראותך כיצד נכפול שרש אחד באחר פשוט |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *{{#annot:√4×√9|737|NYp7}}Suppose you wish to multiply a root of four by a root of nine. |
| + | :<math>\scriptstyle\sqrt{4}\times\sqrt{9}</math> | ||
| + | |style="text-align:right;"|נניח שרצית לכפול שרש ארבעה בשרש תשעה{{#annotend:NYp7}} | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :You should multiply the numbers by each other, which are 4 by 9; the result is 36. |
| + | :<math>\scriptstyle{\color{blue}{4\sdot9=36}}</math> | ||
| + | |style="text-align:right;"|הנה צריך שתכפול המספרי' זה על זה שהם ד' על ט' ועלה ל"ו | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :The root of 36 is the root of the product of a root of 4 by a root of 9. The root of 36 is six. |
| + | :<math>\scriptstyle{\color{blue}{\sqrt{4}\times\sqrt{9}=\sqrt{36}=6}}</math> | ||
| + | |style="text-align:right;"|ושרש זה הל"ו הוא שרש <s>כפל</s> {{#annot:term|241,1241|UxpB}}העולה מכפל{{#annotend:UxpB}} שרש ד' בשרש ט‫'<br> | ||
| + | ושרש הל"ו האלה הוא ששה | ||
|- | |- | ||
| − | | | + | |The proof of this example: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והנה ה{{#annot:term|198|IfyF}}ראיה ל{{#annotend:IfyF}}דמיון |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Since the root of 4 is 2 and the root of 9 is 3, as you have seen above. |
| + | :<math>\scriptstyle{\color{blue}{\sqrt{4}=2}}</math>; <math>\scriptstyle{\color{blue}{\sqrt{9}=3}}</math> | ||
| + | |style="text-align:right;"|בהיות כי שרש ד' הוא ב' ושרש ט' הוא ג' כאשר הראית למעלה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :So, when the root of 4, which is 2, is multiplied by the root of 9, which is 3, i.e. 2 times 3; the result is 6, which is indeed the root of 36. |
| + | :<math>\scriptstyle{\color{blue}{\sqrt{4}\times\sqrt{9}=2\sdot3=6=\sqrt{36}}}</math> | ||
| + | |style="text-align:right;"|הנה כאשר {{#annot:term|358,2025|l2on}}יוכה{{#annotend:l2on}} שרש ד' שהוא ב' בשרש ט' שהוא ג' דהינו ב' פעמי' ג' יעשה ו' שהוא באמת שרש ל"ו | ||
|- | |- | ||
| − | | | + | |I have already taught you previously that every number that is multiplied by itself is the root of that product. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{OliveGreen}{\sqrt{a\sdot a}=a}}</math> |
| + | |style="text-align:right;"|בהיות כי <sup>כבר</sup> למדתיך בעבר שכל מספר אשר יוכה בעצמו הנה הוא שרש מ{{#annot:term|241,1241|jjjX}}העולה מן ההכאה{{#annotend:jjjX}} | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Therefore, six is indeed the root of its product by itself, which is 36. |
| + | :<math>\scriptstyle{\color{blue}{6=\sqrt{6\sdot6}=\sqrt{36}}}</math> | ||
| + | |style="text-align:right;"|ולכן ששה הוא היטב שרש מהכאתו בעצמו שהוא ל"ו | ||
|- | |- | ||
| − | | | + | |According to this example, in which I have taught you to multiply one root by the other, for two numbers that have a known and visible root, since when multiplying denominated numbers, or roots [= the radicands], one by the other, the root of the product should be extracted, so also one should multiply the root of any number by another root and then extract the root of the product, be it visible or concealed. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{OliveGreen}{\sqrt{a}\times\sqrt{b}=\sqrt{a\sdot b}}}</math> |
| + | |style="text-align:right;"|ועל זה הדמיון אשר למדתיך להכות שרש אחד באחר באלו שני מספרי' אשר להם שרש <s>גכ</s> ידוע ונגלה<br> | ||
| + | כי {{#annot:term|185,1255|5mJU}}בהכות ה{{#annotend:5mJU}}מספרי' הנקובים או נאמ' שרשים האחד באחר צריך לקחת שרש {{#annot:term|876,1241|0sNH}}העולה מ{{#annotend:0sNH}}ההכאה ההיא<br> | ||
| + | כן ג"כ צריך לכפול שרש כל מספר בשרש אחר ולקחת שרש העולה מן ההכאה רצוני מ{{#annot:term|156,1229|URB9}}הכפלתו{{#annotend:URB9}} גלוי יהיה או לא גלוי | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :A root of 4 multiplied by a root of 9 yields a root of 36. |
| + | |style="text-align:right;"|שרש ד' פעם שרש ט' עושה שרש מל"ו | ||
| + | |} | ||
| + | {| | ||
|- | |- | ||
| | | | ||
| − | + | ||
| − | + | ==== <span style=color:Green>Multiplication of a Root of a Number by a Number</span> ==== | |
| | | | ||
| − | |||
|- | |- | ||
| − | | | + | |If you want to multiply a root of a number by a number |
| − | |style="text-align:right;"| | + | |style="width:45%;text-align:right;"|<big>ואם</big> רצית לכפול שרש מספר במספר |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *{{#annot:√6×3|737|aKDT}}Suppose you wish to multiply a root of six by three. |
| + | :<math>\scriptstyle\sqrt{6}\times3</math> | ||
| + | |style="text-align:right;"|נניח שרצית לכפול <s>ו'</s> שרש ששה בשלשה{{#annotend:aKDT}} | ||
|- | |- | ||
| − | | | + | |Convert the number into a root: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אז {{#annot:term|1071,1431|ET7N}}תשיב המספר אל שרש{{#annotend:ET7N}} |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :That is 3, which is a root of nine. |
| + | :<math>\scriptstyle{\color{blue}{3=\sqrt{9}}}</math> | ||
| + | |style="text-align:right;"|דהיינו ג' שהוא שרש תשעה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :We then say that we should multiply a root of six by a root of nine. |
| + | :<math>\scriptstyle{\color{blue}{\sqrt{6}\times3=\sqrt{6}\sdot\sqrt{9}}}</math> | ||
| + | |style="text-align:right;"|ונאמר אם כן שאנחנו צריכי' להכות שרש ששה בשרש תשעה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Its instruction is to multiply 6 by 9, which yields 54. |
| + | :<math>\scriptstyle{\color{blue}{6\sdot9=54}}</math> | ||
| + | |style="text-align:right;"|אשר למודו הוא להכות ו' על ט' שעושה נ"ד | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :The root of 54 is the product of a root of six by three and this itself is a root of six multiplied by a root of nine. |
| + | :<math>\scriptstyle{\color{blue}{\sqrt{6}\times3=\sqrt{6}\sdot\sqrt{9}=\sqrt{54}}}</math> | ||
| + | |style="text-align:right;"|ושרש נ"ד הוא {{#annot:term|156,1230|a8tg}}כפל{{#annotend:a8tg}} שרש ששה בשלשה<br> | ||
| + | והוא הוא שרש ששה מוכה בשרש תשעה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :So, the root of 54 is hidden, therefore we express it as a hidden, invisible number. |
| + | |style="text-align:right;"|והנה שרש נ"ד הוא נעלם וכן נבטא בו בנעלם ובמספר בלתי גלוי | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :I mean, we say: a root of 54 is the result of the multiplication of a root of six by the number three. |
| + | :<math>\scriptstyle{\color{blue}{\sqrt{6}\times3=\sqrt{54}}}</math> | ||
| + | |style="text-align:right;"|רצוני לומר <sup>אמרנו</sup> <s>ב</s>שרש נ"ד עולה הכאת שרש ששה במספרי' שלשה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :A root of 6 multiplied by 3, i.e. a root of 6 multiplied by a root of 9, yields a root of 54. |
| + | |style="text-align:right;"|שרש ו' מוכה בג' דהיינו שרש ו' מוכה בשרש ט' עושה שרש נ"ד | ||
| + | |} | ||
| + | {| | ||
|- | |- | ||
| | | | ||
| − | + | ||
| − | + | ==== <span style=color:Green>Multiplication of a Root of a Number by a Number and a Root of a Number</span> ==== | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| | | | ||
| − | |style="text-align:right;"| | + | |- |
| − | + | |If you wish to multiply a root of a number by a number and by a root of a number: | |
| − | + | |style="width:45%;text-align:right;"|‫<ref>2r</ref><big>ואם</big> רצית לכפול שרש מספר <sup>במספר</sup> ובשרש מספר | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *{{#annot:√5×(√7+4)|737|ek9n}}Suppose you wish to multiply a root of 5 by a root of 7 plus 4. |
| + | :<math>\scriptstyle\sqrt{5}\times\left(\sqrt{7}+4\right)</math> | ||
| + | |style="text-align:right;"|נניח שרצית לכפול שרש ה' בשרש ז' וד‫'{{#annotend:ek9n}} | ||
| + | |- | ||
| + | |You should convert now the number into a root: | ||
| + | |style="text-align:right;"|עתה אתה צריך להשיב המספר לשרש | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :That is, 4, which is a root of 16. |
| − | + | :<math>\scriptstyle{\color{blue}{4=\sqrt{16}}}</math> | |
| − | + | |style="text-align:right;"|דהיינו ד' שהוא שרש לי"ו | |
| − | |||
| − | שרש | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :We then say that we need to multiply a root of 5 by a root of 16 and by a root of 7. |
| − | + | :<math>\scriptstyle{\color{blue}{\sqrt{5}\times\left(4+\sqrt{7}\right)=\sqrt{5}\times\left(\sqrt{16}+\sqrt{7}\right)}}</math> | |
| − | + | |style="text-align:right;"|ונאמ' א"כ שצריך שנכה שרש ה' בשרש י"ו ובשרש ז‫' | |
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :We should multiply 5 by 16; the result is 80. |
| + | :<math>\scriptstyle{\color{blue}{5\sdot16=80}}</math> | ||
| + | |style="text-align:right;"|כי עתה צריך לנו לעשות ה' מוכה בי"ו עושה פ‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :For, the root of 80 is the product of a root of 5 by 4. Keep this root of 80 as one part of the multiplication. |
| − | + | :<math>\scriptstyle{\color{blue}{\sqrt{5}\sdot\sqrt{16}=\sqrt{80}}}</math> | |
| − | + | |style="text-align:right;"|כי שרש פ' עולה הכאת שרש ה' <s>בשרש ד'</s> <sup>בד'</sup> אשר שרש פ' זה {{#annot:term|459,1237|mGTf}}תשמור ל{{#annotend:mGTf}}חלק אחד מהכפל | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Then, multiply a root of 5 by the other part, which is a root of 7: |
| − | + | :<math>\scriptstyle{\color{blue}{\sqrt{5}\sdot\sqrt{7}}}</math> | |
| − | + | |style="text-align:right;"|ואח"כ תכפול שרש ה' <s>בחלק האחר מהכפל ואחר תכה שרש ה'</s> בחלק האחר שהוא שרש ז‫' | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | = | + | :That is, you should multiply 5 by 7; the result is 35. |
| − | + | :<math>\scriptstyle{\color{blue}{5\sdot7=35}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|דהיינו שאתה צריך לכפול ה' בז' ועושה ל"ה |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :The root of 35 is a root of 5 multiplied by 7. |
| − | + | :<math>\scriptstyle{\color{blue}{\sqrt{35}=\sqrt{5\sdot7}}}</math> | |
| − | + | |style="text-align:right;"|ושרש זה הל"ה עושה שרש ה' מוכה בז‫' | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | = | + | :Add the root of 35 to the root you kept, which is a root of 80; you get that when multiplying a root of 5 by 4 plus a root of 7, the result is a root of 80 plus a root of 35. |
| − | + | :<math>\scriptstyle{\color{blue}{\sqrt{5}\times\left(4+\sqrt{7}\right)=\sqrt{80}+\sqrt{35}}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אשר שרש ל"ה תשים אצל השרש אשר שמרת הוא שרש פ‫'<br> |
| + | ו{{#annot:term|875,1575|y4WU}}יגיע לידך כי{{#annotend:y4WU}} בהכות שרש ה' בד' ושרש ז' עושה <s>ו</s>שרש פ' ושרש ל"ה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :A root of 5 multiplied by 4 plus a root of 7 yields a root of 80 plus a root of 35. |
| − | + | |style="text-align:right;"|שרש ה' מוכה בד' ושרש ז' עושה שרש פ' ושרש ל"ה | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|} | |} | ||
{| | {| | ||
|- | |- | ||
| | | | ||
| − | |||
| − | |style="text-align:right;"| | + | ==== <span style=color:Green>Multiplication of a Number minus a Root by a Root</span> ==== |
| + | | | ||
| + | |- | ||
| + | |If you wish to multiply a number minus a root by a root: | ||
| + | |style="width:45%;text-align:right;"|<big>ואם</big> רצית לכפול מספר פחות שרש בשרש | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *{{#annot:√3×(6-√8)|737|tW5A}}Suppose you wish to multiply a root of 3 by six minus a root of 8. |
| − | + | :<math>\scriptstyle\sqrt{3}\times\left(6-\sqrt{8}\right)</math> | |
| − | + | |style="text-align:right;"|נניח שרצית לכפול שרש ג' בששה פחות שרש ח‫'{{#annotend:tW5A}} | |
| − | + | |- | |
| − | + | |You should now convert the number into a root: | |
| − | + | |style="text-align:right;"|אתה צריך עתה להשיב המספר לשרש | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | = | + | :That is, 6; you get a root of 36. |
| − | + | :<math>\scriptstyle{\color{blue}{6=\sqrt{36}}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|דהיינו ו' ויהיה לך שרש מל"ו |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :We say then that we should multiply a root of 3 by a root of 36 minus a root of 8. |
| − | + | :<math>\scriptstyle{\color{blue}{\sqrt{3}\times\left(6-\sqrt{8}\right)=\sqrt{3}\times\left(\sqrt{36}-\sqrt{8}\right)}}</math> | |
| − | + | |style="text-align:right;"|ונאמ' א"כ שאנחנו צריכי' לכפול שרש ג' בשרש ל"ו פחות שרש ח‫' | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | צריכי' | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | = | + | :We now need to multiply 3 by 36; the result is 108. |
| − | + | :<math>\scriptstyle{\color{blue}{3\sdot36=108}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|שעתה צריכי' אנו <s>לעשות</s> להכות ג' בל"ו ויעשה ק"ח |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :The root of 108 is the product of the root 3 by the root 36. Keep the root of 108 as one part of the multiplication. |
| − | + | :<math>\scriptstyle{\color{blue}{\sqrt{108}=\sqrt{3}\sdot\sqrt{36}}}</math> | |
| − | + | |style="text-align:right;"|ושרש ק"ח יהיה {{#annot:term|156,1256|23wI}}הכאת{{#annotend:23wI}} שרש ג' בשרש ל"ו אשר זה השרש מק"ח תשמור [ל]חלק אחד מן ההכאה | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | ק | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | ו | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | ל | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | = | + | :Then, multiply a root of 3 by a subtractive root of 8; the result is a root of 24 that should be subtracted from a root of 108. |
| − | + | :<math>\scriptstyle{\color{blue}{\sqrt{3}\sdot\sqrt{8}=\sqrt{24}}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואחר תכה שרש ג' בפחות שרש ח' שעולה שרש כ"ד שצריך להוציא משרש ק"ח |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :A root of 108 minus a root of 24 remain; so when multiplying a root of 3 by six minus a root of 8, the result is a root of 108 minus a root of 24. |
| − | + | :<math>\scriptstyle{\color{blue}{\sqrt{3}\times\left(6-\sqrt{8}\right)=\sqrt{108}-\sqrt{24}}}</math> | |
| − | + | |style="text-align:right;"|ו{{#annot:term|936,1236|tWMC}}ישאר{{#annotend:tWMC}} שרש ק"ח פחות שרש כ"ד<br> | |
| − | + | א"כ בהכות <s>שש</s> שרש ג' בששה פחות שרש ח' יעלה שרש ק"ח פחות שרש כ"ד | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|} | |} | ||
{| | {| | ||
|- | |- | ||
| | | | ||
| − | |||
| − | + | ==== <span style=color:Green>Multiplication of a Number and a Root by a Number and a Root - when the numbers are identical and the roots are identical</span> ==== | |
| + | |||
| + | | | ||
|- | |- | ||
| − | | | + | |If you want to multiply a number and a root by a number and a root: |
| − | |style="text-align:right;"| | + | |style="width:45%;text-align:right;"|<big>עוד</big> אם רצית לכפול מספר ושרש במספר ושרש |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | + | *{{#annot:(3+√5)×(3+√5)|737|gk0X}}Suppose you wish to multiply 3 plus a root of 5 by 3 plus a root of 5. | |
| − | + | :<math>\scriptstyle\left(3+\sqrt{5}\right)\times\left(3+\sqrt{5}\right)</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|נניח שרצית לכפול ג' ושרש ה' בג' ושרש ה‫'{{#annotend:gk0X}} |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :You should multiply first the numbers by each other, which are 3 by 3; the result is 9. |
| − | + | :<math>\scriptstyle{\color{blue}{3\sdot3=9}}</math> | |
| − | + | |style="text-align:right;"|צריך אתה להכות ראשונה המספרי' זה על זה והם ג' על ג' ועולה ט‫' | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | == | + | :Then, multiply the roots by each other, which are a root of 5 by a root of 5; the result is a root of 25; this root is 5. |
| − | + | :<math>\scriptstyle{\color{blue}{\sqrt{5}\sdot\sqrt{5}=\sqrt{25}=5}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אחר כך תכה השרשי' זה על זה שהם שרש ה' בשרש ה' ועולה שרש כ"ה אשר זה השרש הוא ה‫' |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Add 5 to 9; they are 14 numbers. |
| − | + | :<math>\scriptstyle{\color{blue}{9+5=14}}</math> | |
| − | + | |style="text-align:right;"|ותקבץ אלו הה' עם ט' ויהיו י"ד מספרי‫' | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |Then, convert the numbers into a root: |
| − | + | |style="text-align:right;"|אח"כ {{#annot:term|1071,1431|1lg4}}תשיב המספרי' לשרשי‫'{{#annotend:1lg4}} | |
| − | |||
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :I.e. for each of the 3 you have a root of 9. |
| − | + | :<math>\scriptstyle{\color{blue}{3=\sqrt{9}}}</math> | |
| − | + | |style="text-align:right;"|דהיינו כל אחד מהם שהוא ג' ויהיה לך בעד כל אחד מהם שרש ט‫' | |
| − | |||
| − | |||
| − | כל אחד | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | + | :Multiply these roots crosswise by the root of 5, meaning that you should multiply the root of 5 by the root of 9, then again the root of 5 by the root of 9; you get a root of 45 for each of these products and these two roots are equal to each other. | |
| − | + | |style="text-align:right;"|ואלו השר<sup>ש</sup>ים {{#annot:term|2521,2522|Msif}}תכה בשתי וערב{{#annotend:Msif}} על שרש ה' רצוני שצריך אתה להכות שרש ה' בשרש ט' ואח"כ ג"כ שרש ה' בשרש ט' ויעלה לך בעד כל אחת מה{{#annot:term|156,1256|DpJh}}הכאות{{#annotend:DpJh}} שרש מ"ה ושני השרשי' האלו הם {{#annot:term|461,1247|oP1J}}שוים זה לזה{{#annotend:oP1J}} | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Summed together they are four times the root of 45, which is a root of 180. |
| − | + | |style="text-align:right;"|ו{{#annot:term|178,1841|7s8E}}מקובצי' יחד{{#annotend:7s8E}} יעשו שרש מארבעה דמיונם דהיינו שרש מארבעה פעמים <s>כ"ה</s> מ"ה שעולה שרש מק"פ | |
| − | + | |- | |
| − | + | |colspan=2| | |
| − | + | ::<math>\scriptstyle{\color{blue}{\left(3\sdot\sqrt{5}\right)+\left(\sqrt{5}\sdot3\right)=\left(\sqrt{9}\sdot\sqrt{5}\right)+\left(\sqrt{5}\sdot\sqrt{9}\right)=\sqrt{45}+\sqrt{45}=\sqrt{4\sdot45}=\sqrt{180}}}</math> | |
| − | + | |- | |
| − | + | |Know that for every two roots that are summed together, if they are equal, they yield four times their root. | |
| − | + | :<math>\scriptstyle{\color{OliveGreen}{\sqrt{a}+\sqrt{a}=\sqrt{4a}}}</math> | |
| − | + | |style="text-align:right;"|<big>ודע</big> כי כל שני שרשי' מקובצי' יחד בהיותם שוים יעשו שרש לארבעה דמיונם | |
| − | + | |- | |
| − | |} | + | |From this you seen the proof of the teaching of the addition of unequal roots. |
| − | {| | + | |style="text-align:right;"|ומזה תראה ה{{#annot:term|198,1898|1r4t}}מופת ב{{#annotend:1r4t}}למוד חבור השרשי' {{#annot:term|962,1247|fpHc}}הבלתי שוים{{#annotend:fpHc}} |
|- | |- | ||
| | | | ||
| − | == | + | :Now, add the root of 180 to the two other parts we summed together that are 14; you have 14 and a root of 180 and this is the result of 3 plus a root of 5 multiplied by 3 plus a root of 5. |
| − | + | :<math>\scriptstyle{\color{blue}{\left(3+\sqrt{5}\right)\times\left(3+\sqrt{5}\right)=14+\sqrt{180}}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ועתה תקבץ זה השרש מק"פ עם שני החלקי' האחרים אשר קבצנו יחד והיו י"ד<br> |
| + | ויהיה לך י"ד ושרש ק"פ וכן {{#annot:term|875,1240|Aiix}}עולה{{#annotend:Aiix}} ג' ושרש ה' מוכה בג' ושרש ה‫' | ||
| + | |- | ||
| + | |Know that every root of a number multiplied by another identical to it, is the same as saying "its product by itself"; it yields that same number itself, by which the root is denominated [= the radicand]. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\sqrt{a}\times\sqrt{a}=a}}</math> | ||
| + | |style="text-align:right;"|<big>ודע</big> כי כל שרש מספר מוכה באחר שוה לו הוא שוה לאמרך הכאה זו בעצמה ועושה המספר ההוא עצמו אשר ממנו נקרא שרש | ||
| + | |- | ||
| + | |Therefore, when you multiply 3 plus a root of 5 by 3 plus a root of 5, or any other given identical roots, you only need to add the number by which the root is denominated [= the radicand] to the product of the numbers. | ||
| + | |style="text-align:right;"|ולזה תכה ג' ושרש ה' בג' ושרש ה' או שרשי' אחרים שיותנו שוים אין לך רק לקבץ על הכאת המספרים המספר אשר שרשו נקוב | ||
| + | |- | ||
| + | |If the numbers are the same, sum them together, then convert their sum into a root, and multiply this root by one of the roots of the product. | ||
| + | |style="text-align:right;"|ואם המספרים שוים תקבצם יחד ואחר תשיב ‫<ref>2v</ref>הכלל ההוא אל שרש ותכה השרש ההוא <s>בשרש אחד</s> באחד מהשרשי' אשר בהכאה | ||
| + | |- | ||
| + | |Add the root of this product to the sum of the numbers, meaning to the product of the summed numbers. | ||
| + | |style="text-align:right;"|ושרש זאת ההכאה תקבץ עם המספרי' ש{{#annot:term|178,1841|Nbrj}}קובצו יחד{{#annotend:Nbrj}}<br> | ||
| + | רצוני על הכאת המספרי' ש{{#annot:term|178,1841|FwN9}}קובצו ל{{#annotend:FwN9}}שרש | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\left(a+\sqrt{b}\right)\times\left(a+\sqrt{b}\right)=\left[a\sdot\left(a+\sqrt{b}\right)\right]+b=\left(a\sdot a\right)+\sqrt{\left(a+a\right)^2\sdot b}+b}}</math> | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|עוד | + | *Example: suppose you wish to multiply again 3 plus a root of 5 by 3 plus a root of 5. |
| − | + | :<math>\scriptstyle\left(3+\sqrt{5}\right)\times\left(3+\sqrt{5}\right)</math> | |
| − | + | |style="text-align:right;"|<big>והנה</big> המשל נניח שרצונך עוד להכות ג' ושרש ה' בג' ושרש ה‫' | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | = | + | :You should multiply the numbers by each other. |
| − | + | :<math>\scriptstyle{\color{blue}{3\sdot3=9}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אתה צריך להכות המספרי' יחד דהיינו ג' בג' ועושה ט‫' |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Then, add the number by which the root is denominated [= the radicand], which is 5, to them; you have 14. Keep it. |
| − | + | :<math>\scriptstyle{\color{blue}{9+5=14}}</math> | |
| − | + | |style="text-align:right;"|אח"כ תקבץ עמהם המספר {{#annot:term|1073,2300|RNkz}}הנקוב אשר לו השרש{{#annotend:RNkz}} והוא ה' ויהיה לך י"ד ו{{#annot:term|459,1237|Tim1}}תשמרם{{#annotend:Tim1}} | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | המספר | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |} | ||
| − | { | ||
|- | |- | ||
| | | | ||
| − | + | :Sum the numbers together, meaning 3 and 3; they are 6. | |
| − | + | |style="text-align:right;"|אח"כ תקבץ המספרי' יחד רצוני ג' וג' ויהיו ו‫' | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Convert 6 into a root; you have a root of 36. |
| − | + | |style="text-align:right;"|ואלו הו' {{#annot:term|1071,1431|OVQV}}תשיב אל שרש{{#annotend:OVQV}} ויהיה לך שרש ל"ו | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | == | + | :Multiply the root of 36 by a root of 5; the result is a root of 180. |
| − | + | :<math>\scriptstyle{\color{blue}{\left(3+3\right)\sdot\sqrt{5}=6\sdot\sqrt{5}=\sqrt{36}\sdot\sqrt{5}=\sqrt{180}}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ושרש ל"ו זה {{#annot:term|185,1255|QPTS}}תכה ב{{#annotend:QPTS}}שרש ה' ויעלה שרש ק"פ |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Now, add the root of 180 to the 14 you kept; their sum is 14 plus a root of 180 and this is the product of 3 plus a root of 5 by 3 plus a root of 5. |
| − | על | + | :<math>\scriptstyle{\color{blue}{\left(3+\sqrt{5}\right)\times\left(3+\sqrt{5}\right)=14+\sqrt{180}}}</math> |
| − | + | |style="text-align:right;"|ועתה תקבץ שרש ק"פ על י"ד אשר שמרת ויהיה כללם י"ד ושרש ק"פ וככה תעלה הכאת ג' ושרש ה' בג' ושרש ה‫' | |
| − | + | |- | |
| − | + | |The aforesaid rule is complete. | |
| − | + | |style="text-align:right;"|ונשלם הכלל האמור | |
| − | + | |- | |
| − | + | |I inform you that the three aforementioned methods are also written in the next page. | |
| − | + | |style="text-align:right;"|<big>ואודיעך</big> כי שלש השטות הנזכרו' יהיו כתובות ג"כ <s>בקל</s> בעלה <s>ב</s>הנמשך אחר זה | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |In order to establish this rule and the one that follows in the next page we brought them here. |
| − | + | |style="text-align:right;"|ולתקן זה הכלל ואשר ימשך אחריו בדף הנמשך הנחנוהו הנה | |
| − | |||
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :The product of 3 plus a root of 5 by 3 plus a root of 5 is 14 numbers and a root of 180. |
| − | + | :<math>\scriptstyle{\color{blue}{\left(3+\sqrt{5}\right)\times\left(3+\sqrt{5}\right)=14+\sqrt{180}}}</math> | |
| − | + | |style="text-align:right;"|והנה לכפול ג' ושרש ה' בג' ושרש ה' יעלה י"ד מספרים ושרש ק"פ | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|} | |} | ||
{| | {| | ||
|- | |- | ||
| | | | ||
| − | |||
| − | |style="text-align:right;"| | + | ==== <span style=color:Green>Multiplication of a Number and a Root by a Number and a Root</span> ==== |
| + | | | ||
| + | |- | ||
| + | |I want to show you also another rule common to all multiplications of numbers and roots either equal or unequal: | ||
| + | |style="width:45%;text-align:right;"|<big>עוד</big> רצוני להראותך כלל אחר משותף לכל כפל ממספרי' ושרשי' שוים יהיו או בלתי שוים | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *{{#annot:(3+√4)×(4+√9)|737|QhWc}}Suppose you wish to multiply 3 plus a root of 4 by 5 plus a root of 9. |
| − | + | :<math>\scriptstyle\left(3+\sqrt{4}\right)\times\left(5+\sqrt{9}\right)</math> | |
| − | + | |style="text-align:right;"|ונניח כי בקשת לכפול ג' ושרש ד' בה' ושרש ט‫'{{#annotend:QhWc}} | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |Although you will find this rule in this book below also, since the numbers of the roots are visible, discrete in a foreign language, we present it here. |
| − | + | |style="text-align:right;"|גם כי תמצא זה הכלל בזה הספר בהמשך אבל מפני כי מספרי השרשים הם גלויים דיסקריטי בלעז נניחהו הנה | |
| − | |||
| − | |style="text-align:right;"| | ||
|- | |- | ||
| − | | | + | |First, we multiply the numbers by each other. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וראשונה נכפול המספרי' זה נגד זה |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | = | + | :Meaning 3 by 5; the result is 15. Keep it. |
| − | + | :<math>\scriptstyle{\color{blue}{3\sdot5=15}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|רצוני ג' בה' ועולה ט"ו ושמרהו |
|- | |- | ||
| − | | | + | |Then, multiply the numbers crosswise by the roots, while converting the numbers into roots: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אח"כ תכפול המספרי' בשרשי' בשתי וערב {{#annot:term|1071,1431|uLzJ}}בהשיב{{#annotend:uLzJ}} תמיד המספרי' לשרשי‫' |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :So, we multiply 3 by a root of 9; the result is a root of 81, because, when converting 3 into a root it becomes a root of 9 and when multiplying a root of 9 by a root of 9, the result is 9, which is indeed a root of 81. |
| − | + | :<math>\scriptstyle{\color{blue}{3\sdot\sqrt{9}=\sqrt{9}\sdot\sqrt{9}=\sqrt{81}=9}}</math> | |
| − | + | |style="text-align:right;"|וא"כ נכה ג' בשרש ט' ויעלה שרש פ"א מפני כי בהשיב ג' אל שרש שיהיה שרש ט' ו{{#annot:term|185,1255|IS0U}}הכות{{#annotend:IS0U}} שרש ט' בשרש ט' יעשה ט' שבאמת הוא שרש פ"א | |
| − | שרש | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | = | + | :And vice versa for the other number, i.e. we multiply it by a root of 4; the result is a root of 100. |
| − | + | :<math>\scriptstyle{\color{blue}{5\sdot\sqrt{4}=\sqrt{100}}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכן הוא קונבירסו המספר האחר היינו ה' {{#annot:term|185,1255|Ppcv}}נכהו נגד{{#annotend:Ppcv}} שרש ד' ועושה שרש ק‫' |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Sum these two products with the numbers you kept; they are 15, so you get 15 and a root of 81 plus a root of 100. |
| − | + | :<math>\scriptstyle{\color{blue}{15+\sqrt{81}+\sqrt{100}}}</math> | |
| − | + | |style="text-align:right;"|ואלו שתי ההכאות תקבץ עם המספרי' אשר שמרת והיו ט"ו ויהיו לך ט"ו ושרש פ"א ושרש ק‫' | |
| − | + | |- | |
| − | ההכאות | + | |Now, you should multiply the roots by each other. |
| − | + | |style="text-align:right;"|ועתה צריך אתה לכפול השרשי' האחד על האחר | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | = | + | :Meaning, a root of 4 by a root of 9; you have a root of 36. |
| − | + | :<math>\scriptstyle{\color{blue}{\sqrt{4}\sdot\sqrt{9}=\sqrt{36}}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|רצוני שרש ד' בשרש ט' ויהיה לך שרש ל"ו |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Add it to the former sum; the result is the total product, i.e. 15 plus a root of 81, a root of 100, and a root of 36, which is 40 in a visible number. |
| − | + | :<math>\scriptstyle{\color{blue}{\left(3+\sqrt{4}\right)\times\left(5+\sqrt{9}\right)=15+\sqrt{81}+\sqrt{100}+\sqrt{36}=40}}</math> | |
| − | + | |style="text-align:right;"|ותקבצהו אל הסך הראשון ויצא <sup>לך</sup> בסך כל ה{{#annot:term|241,1229|AM16}}הכפלה{{#annotend:AM16}} דהיינו ט"ו ושרש פ"א ושרש ק' ושרש ל"ו<br> | |
| − | + | שהם במספר גלוי מ‫' | |
| − | + | |- | |
| − | + | |From this rule you can understand all the others whether they involve a visible number or a hidden number. | |
| − | + | |style="text-align:right;"|ומזה הכלל תוכל להבין כל האחרים בין שיהיה להם מספר גלוי או מספר נעלם | |
| − | + | |- | |
| − | + | |For the chapter written below was written first at the end of the next page in the context of - "If you wish to multiply a number minus a root by a number minus a root". | |
| − | + | |style="text-align:right;"|<big>ואודיעך</big> כי זה הפרק הכתו' תחת זה הותחל ליכתב בסוף העלה הנמשך האומר ואם רצית להכות מספר פחות שרש במ[ס]פר פחות שרש | |
| − | + | |- | |
| − | + | |But, since it was not fully written below as it should have been, and that was a mistake, we present it and explain it properly here. | |
| − | + | |style="text-align:right;"|ובעבור שלא נשלם ליכתב בהמשך כאשר היה ראוי להמשיך וזה היה משגה לכן נניחהו הנה מבואר כראוי | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|} | |} | ||
{| | {| | ||
|- | |- | ||
| | | | ||
| − | |||
| − | |style="text-align:right;"| | + | ==== <span style=color:Green>Multiplication of a Number minus a Root by a Number minus a Root</span> ==== |
| + | | | ||
| + | |- | ||
| + | |I say: if you wish to multiply a number minus a root by a number minus a root: | ||
| + | |style="width:45%;text-align:right;"|<big>ואומר</big> אם רצית להכות מספר פחות שרש במספר פחות שרש | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *{{#annot:(3-√5)×(4-√7)|737|0gkX}}Example: if you wish to multiply 3 minus a root of 5 by 4 minus a root of 7. |
| − | + | :<math>\scriptstyle\left(3-\sqrt{5}\right)\times\left(4-\sqrt{7}\right)</math> | |
| − | + | |style="text-align:right;"|{{#annot:term|197,1712|bB8m}}נעשה הדמיון ש{{#annotend:bB8m}}רצית לכפול ג' פחות שרש ה' בד' פחות שרש ז‫'{{#annotend:0gkX}} | |
| − | + | |- | |
| − | + | |First, you should multiply the numbers by each other: | |
| − | + | |style="text-align:right;"|אתה צריך ראשונה לכפול המספרי' זה על זה | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | פחות שרש | ||
| − | |||
| − | פחות שרש | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | == | + | :They are 3 by [4]; the result is 12. Keep it. |
| − | + | :<math>\scriptstyle{\color{blue}{3\sdot4=12}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והם ג' בו' ועולה י"ב ושמרם |
| + | |- | ||
| + | |Then, multiply the numbers by the subtractive roots crosswise; the result is a subtractive root: | ||
| + | |style="text-align:right;"|אח"כ {{#annot:term|2521,2522|tlny}}תכפול המספרי' בשתי וערב{{#annotend:tlny}} בשביל השרשי' שהם {{#annot:term|789,2032|wD9K}}פוחתי'{{#annotend:wD9K}} ‫<ref>3r</ref>ומה שיעלה יהיה שרש {{#annot:term|789,1366|5kZw}}פחות{{#annotend:5kZw}} | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :So, multiply 3 by a subtractive root of 7; the result is a subtractive root of 63. |
| − | + | :<math>\scriptstyle{\color{blue}{3\sdot\left(-\sqrt{7}\right)=-\sqrt{63}}}</math> | |
| − | + | |style="text-align:right;"|לכן תכה ג' <sup>ב</sup>פחות שרש ז' ועולה שרש ס"ג {{#annot:term|789,2032|i18t}}פוחת{{#annotend:i18t}} | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |} | ||
| − | { | ||
|- | |- | ||
| | | | ||
| − | = | + | :Then, multiply 4 by a subtractive root of 5; the result is a subtractive root of 80. |
| − | + | :<math>\scriptstyle{\color{blue}{4\sdot\left(-\sqrt{5}\right)=-\sqrt{80}}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואחר תכפול ד' בפחות שרש ה' ועולה שורש פ' פוחת |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Sum the two subtractive roots together; you have a subtractive root of 63 plus a subtractive root of 80. |
| − | + | :<math>\scriptstyle{\color{blue}{\left(-\sqrt{63}\right)+\left(-\sqrt{80}\right)=-\sqrt{63}-\sqrt{80}}}</math> | |
| − | + | |style="text-align:right;"|אח"כ תקבץ שני אלו השרשי' יחד שהם פוחתי' ויהיה לך שרש ס"ג פוחת ושרש פ' פוחת | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | == | + | :These two subtractive roots should be subtracted from the additive product. |
| − | + | |style="text-align:right;"|ואלו שני שרשי' {{#annot:term|789,2032|trCC}}הפוחתים{{#annotend:trCC}} צריך להוציאם מן סכום ההכפלה שעושה יותר | |
| − | |style="text-align:right;"| | + | |- |
| + | |Now, know that you should multiply the two subtractive roots by each other; the result is an additive root. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\left(-\sqrt{}\right)\times\left(-\sqrt{}\right)=+\sqrt{}}}</math> | ||
| + | |style="text-align:right;"|ועתה דע כי צריך אתה לכפול שני השרשי' הפוחתים זה על זה ויעשה שרש יותר | ||
| + | |- | ||
| + | |Add this product to the number resulting from the multiplication of the numbers by each other. | ||
| + | |style="text-align:right;"|וזאת ההכפלה {{#annot:term|178,1206|2UjC}}תוסיף על{{#annotend:2UjC}} {{#annot:term|876,1233|2AKq}}המספר היוצא מ{{#annotend:2AKq}}הכאת המספרי' זה על זה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :So, multiply [a subtractive root of] 5 by a subtractive root of 7; the result is an additive root of 35. |
| − | + | :<math>\scriptstyle{\color{blue}{{\color{red}{\left(-\sqrt{5}\right)}}\sdot\left(-\sqrt{7}\right)=\sqrt{35}}}</math> | |
| − | + | |style="text-align:right;"|א"כ תכה ה' בשרש ז' פוחת ועושה שרש ל"ה יותר | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | + | :Add this root to the numbers, i.e. to 12; you have 12 plus a root of 35. | |
| − | + | :<math>\scriptstyle{\color{blue}{12+\sqrt{35}}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וזה השרש תוסיף על {{#annot:term|241,1229|vPsa}}הכפלת ה{{#annotend:vPsa}}מספרי' דהיינו על י"ב ויהיה לך י"ב ושרש ל"ה |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Now, subtract the two aforementioned subtractive roots, which are a root of 63 and a root of 80, from the additive product; you get 12 plus a root of 35, minus a root of 63, minus a root of 80, and this is the product of 3 minus a root of 5 by 4 minus a root of 7. |
| − | + | :<math>\scriptstyle{\color{blue}{\left(3-\sqrt{5}\right)\times\left(4-\sqrt{7}\right)=12+\sqrt{35}-\sqrt{63}-\sqrt{80}}}</math> | |
| − | + | |style="text-align:right;"|עתה תוציא שני השרשי' שאמרנו קודם שהם פוחתי' והם שרש ס"ג ושרש פ' מסכום ההכאה שהיה יותר ויהיה לך י"ב ושרש ל"ה פוחת שרש ס"ג ופוחת שרש פ' וככה עולה הכאת ג' פוחת שרש ה' בד' פוחת שרש ז‫' | |
| − | + | |- | |
| − | + | |Know that the result is the same whether starting by multiplying the roots, then the numbers, or starting with the numbers, then the roots. | |
| − | + | |style="text-align:right;"|<big>ודע</big> כי שוה <sup>יצא</sup> הדבר להתחיל בהכאת השרשים ולהשלים במספרי' כמו בהתחיל במספרי' ולהשלים בשרשי‫' | |
| − | + | |- | |
| − | + | |Remember always to sum the additive products together, before starting to subtract a number or a root from any product, in order to have a true understanding of the stated multiplication, or others that will be carried out. | |
| − | + | |style="text-align:right;"|וזכור לעולם להוסיף עליו ההכאות שעושות יותר יחד קודם שתתחיל להוציא המספר או <sup>איזה</sup> <s>ב</s>שרש מאיזו הכאה כדי שתהיה לך הבנה אמתית יותר בהכפלה האמורה ובאחרות שיקרו ושיוכלו להגיע | |
| − | |||
| − | |||
| − | שרש | ||
| − | |||
| − | ודע כי | ||
| − | |||
| − | |||
| − | | | ||
| − | |||
|- | |- | ||
| | | | ||
| − | == | + | :3 minus a root of 5 by 4 minus a root of 7 yields 12 plus a root of 35, minus a root of 63, minus a root of 80. |
| − | + | :<math>\scriptstyle{\color{blue}{\left(3-\sqrt{5}\right)\times\left(4-\sqrt{7}\right)=12+\sqrt{35}-\sqrt{63}-\sqrt{80}}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ג' פחות שרש ה' בד' פחות שרש ז' עושה י"ב ושרש ל"ה פחות שרש ס"ג ופחות שרש פ‫' |
| + | |- | ||
| + | |I also want to show you another rule common to all [types of] multiplications of numbers and roots either equal or unequal: | ||
| + | |style="text-align:right;"|<big>עוד</big> רצוני להראותך כלל אחר משותף לכל כפל <sup>בין</sup> יהיה שוה ממספרי' ומשרשי' או לא יהיה שוה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *{{#annot:(3+√5)×(4+√7)|737|SjVv}}Suppose you wish to multiply 3 plus a root of 5 by 4 plus a root of 7. |
| − | + | :<math>\scriptstyle\left(3+\sqrt{5}\right)\times\left(4+\sqrt{7}\right)</math> | |
| − | + | |style="text-align:right;"|נניח שרצונך לכפול ג' ושרש ה' בד' ושרש ז‫'{{#annotend:SjVv}} | |
| − | + | |- | |
| − | + | |First, you should multiply the numbers by each other: | |
| − | + | |style="text-align:right;"|אתה צריך ראשונה לכפול המספרים יחד | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | = | + | :I.e. 3 by 4; the result is 12. |
| − | + | :<math>\scriptstyle{\color{blue}{3\sdot4=12}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|דהיינו ג' בד' ועולה י"ב |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Then, multiply the numbers by the roots crosswise, while always converting the numbers into roots: |
| − | + | |style="text-align:right;"|אח"כ תכפול המספרי' בשרשים בשתי וערב בהשיב תמיד המספרי' לשרשים | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | = | + | :So, multiply 3 by a root of 7; the result is a root of 63. |
| − | + | :<math>\scriptstyle{\color{blue}{3\sdot\sqrt{7}=\sqrt{63}}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|לכן תכפול ג' בשרש ז' שעולה שרש ס"ג |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Then, multiply 4 by a root of 5; the result is a root of 80. |
| − | + | :<math>\scriptstyle{\color{blue}{4\sdot\sqrt{5}=\sqrt{80}}}</math> | |
| − | + | |style="text-align:right;"|ואח"כ תכפול ד' בשרש ה' עולה שרש פ‫' | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | = | + | :Add these two roots to the number you kept, which is 12; you get 12 plus a root of 63 and a root of 80. |
| − | + | :<math>\scriptstyle{\color{blue}{12+\sqrt{63}+\sqrt{80}}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ושני אלו השרשי' תקבץ אל המספרי' אשר שמרת והיו י"ב ויהיה לך י"ב ושרש ס"ג ושרש פ‫' |
| + | |- | ||
| + | |Now, multiply the two roots by each other: | ||
| + | |style="text-align:right;"|ועתה תכפול השרשים זה על זה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Meaning, a root of 5 by a root of 7; the result is a root of 35. |
| − | + | :<math>\scriptstyle{\color{blue}{\sqrt{5}\sdot\sqrt{7}=\sqrt{35}}}</math> | |
| − | + | |style="text-align:right;"|רצוני שרש ה' בשרש ז' ועולה שרש ל"ה | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | שרש | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | == | + | :Add it to the former sum; you get the total product: 12 plus a root of 63, a root of 80, and a root of 35. |
| − | + | :<math>\scriptstyle{\color{blue}{\left(3+\sqrt{5}\right)\times\left(4+\sqrt{7}\right)=12+\sqrt{63}+\sqrt{80}+\sqrt{35}}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וזה {{#annot:term|178,1165|1jU5}}תחבר אל{{#annotend:1jU5}} הסכום הראשון ויהיה לך סך <s>ה</s>כל הכפל י"ב ושרש ס"ג ושרש פ' ושרש ל"ה |
| + | |- | ||
| + | |Know that the result is the same whether starting by multiplying the roots, or starting with the numbers. | ||
| + | |style="text-align:right;"|<big>ודע</big> כי ככה ישוה <s>לכפול</s> בתחלת ה{{#annot:term|156,1229|qtuD}}הכפלה{{#annotend:qtuD}} להתחיל <sup>לכפול</sup> מהשרשי' כמו להתחיל מן המספרים | ||
| + | |- | ||
| + | |Know also that multiplying one of the numbers by the number and the root of the other term, then multiplying the root given in the term of that number by the number and the root of the other term, and summing all the products together - is the same as the multiplication in the ways mentioned above. | ||
| + | |style="text-align:right;"|<big>עוד</big> דע כי ככה שוה לכפול אחד מהמספרי' במספר אשר בצד האחר ובשרש ואחר תכפול השרש המונח מהמספר במספר ובשרש מהצד האחר ואח"כ תקבץ כל דבר יחד כמו בכל אחד מהאופני' הנזכרי' בזה האופן בכפול הכפל הכתו' למעלה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Now, multiply 3 by 4 and a root of 7; the result is 12 and a root of 63. |
| − | + | :<math>\scriptstyle{\color{blue}{3\sdot\left(4+\sqrt{7}\right)=12+\sqrt{63}}}</math> | |
| − | + | |style="text-align:right;"|תכפול עתה ג' בד' ושרש ז' ועולה י"ב ושרש ס"ג | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | ושרש | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | = | + | :Then, multiply the root given with the number, which is a root of 5, by 4 and a root of 7; the result is a root of 80 and a root of 35; and the mentioned multiplication is complete. |
| − | + | :<math>\scriptstyle{\color{blue}{\sqrt{5}\sdot\left(4+\sqrt{7}\right)=\sqrt{80}+\sqrt{35}}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואחר תכפול השרש המונח מהמספר והוא שרש ה' בד' ושרש ז' עולה שרש פ' ושרש ל"ה ותהיה נשלמת ההכפלה הנזכרת |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :3 plus a root of 5 by 4 plus a root of 7 yields 12 plus a root of 63, a root of 80, and a root of 35. |
| − | + | :<math>\scriptstyle{\color{blue}{\left(3+\sqrt{5}\right)\times\left(4+\sqrt{7}\right)=12+\sqrt{63}+\sqrt{80}+\sqrt{35}}}</math> | |
| − | + | |style="text-align:right;"|ג' ושרש ה' בד' ושרש ז' עולה י"ב ושרש ס"ג ושרש פ' ושרש ל"ה | |
| − | + | |- | |
| − | + | |Also, if you wish to multiply a number minus a root by a number minus a root: | |
| − | + | |style="text-align:right;"|‫<ref>3v</ref><big>והנה</big> עוד אם בקשת לכפול מספר פחות שרש במספר פחות שרש | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | = | + | *{{#annot:(3-√5)×(4-√7)|737|X7yi}}Suppose you wish to multiply 3 minus a root of 5 by 4 minus a root of 7. |
| − | + | :<math>\scriptstyle\left(3-\sqrt{5}\right)\times\left(4-\sqrt{7}\right)</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|נניח שרצית לכפול ג' פחות שרש ה' בד' פחות שרש ז‫'{{#annotend:X7yi}} |
| + | |- | ||
| + | |You should multiply the numbers by each other. | ||
| + | |style="text-align:right;"|אתה צריך לכפול המספרי' זה בזה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :That is 3 by 4; the result is 12. Keep it. |
| − | + | :<math>\scriptstyle{\color{blue}{3\sdot4=12}}</math> | |
| − | + | |style="text-align:right;"|דהיינו ג' בד' ועולה י"ב ושמרהו | |
| − | + | |- | |
| − | + | |Know that this rule is given in the previous page, since it does not continue here. | |
| − | + | |style="text-align:right;"|דע כי זה הכלל הושם בעלה <sup><s>הנמשך</s></sup> שקדם בעבור כי לא נמשך אצל זה | |
| − | + | |- | |
| − | + | |Also, I want to show you that in multiplication of numbers a subtractive by subtractive generates an additive. | |
| − | + | :<math>\scriptstyle{\color{OliveGreen}{\left(-\right)\times\left(-\right)=\left(+\right)}}</math> | |
| − | + | |style="text-align:right;"|<big>עוד</big> רצוני להראותך במספר כי פחות בפחות יעשה יותר בהכפלה | |
| − | + | |- | |
| − | + | |Since every time you wil multiply a subtractive by a subtractive you will clearly see that it yields an additive. | |
| − | + | |style="text-align:right;"|בעבור כי בכל פעם שיזדמן לך לכפול פחות בפחות תראה מבואר שיעשה יותר | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | = | + | :For example: 8 multiplied by 8 yields 64. |
| − | + | :<math>\scriptstyle{\color{blue}{8\sdot8=64}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והנה המשל ח' מוכה בח' עושה ס"ד |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :8 is less than 10 by 2. |
| − | + | :<math>\scriptstyle{\color{blue}{8=10-2}}</math> | |
| − | + | |style="text-align:right;"|וח' הוא ב' פחות מי‫' | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | + | :So, when multiplied by another 8, which is also less than 10 by 2, the result should be the same 64. | |
| − | + | |style="text-align:right;"|ולהכותו בח' אחר שהוא ג"כ פחות מי' ראוי שיעלה כדומה לו ס"ד | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Therefore, we say that 10 minus 2 multiplied by 10 minus 2 yields 64. |
| − | + | :<math>\scriptstyle{\color{blue}{8\sdot8=\left(10-2\right)\sdot\left(10-2\right)=64}}</math> | |
| − | + | |style="text-align:right;"|ולזה נאמ' שי' פחות ב' מוכה בי' פחות ב' ראוי שיעשה ס"ד | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | = | + | :You see that, because when we multiply 10 by 10, it yields 100. |
| − | + | :<math>\scriptstyle{\color{blue}{10\sdot10=100}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וראית זה <sup>כי</sup> בהכותנו י' על י' עושה ק‫' |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :10 multiplied by a subtractive 2 yields a subtractive 20, that is 10 by the 2 that is on the other side. |
| − | + | :<math>\scriptstyle{\color{blue}{10\sdot\left(-2\right)=-20}}</math> | |
| − | + | |style="text-align:right;"|וי' מוכה בב' פחות עושה כ' פחות דהיינו י' בב' שהוא מהצד האחר | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | = | + | :Now, multiply crosswise: 10 that is on the other side by the other 2 that is also subtractiv; it also yields a subtractive 20. |
| − | + | :<math>\scriptstyle{\color{blue}{10\sdot\left(-2\right)=-20}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|עתה תכה בשתי וערב י' שהוא בצד האחר בב' האחר שהוא ג"כ פ<sup>ו</sup>חת עושה ג"כ כ' פוחת |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Sum up these two subtractive products; the result is a subtractive 40. |
| − | + | :<math>\scriptstyle{\color{blue}{\left(-20\right)+\left(-20\right)=-40}}</math> | |
| − | + | |style="text-align:right;"|ועתה תקבץ אלו שתי ההכאות {{#annot:term|789,2032|65AD}}הפוחתות{{#annotend:65AD}} יחד ו{{#annot:term|875,1240|2G63}}יעלו{{#annotend:2G63}} מ' פוחתים | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | יחד | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |} | ||
| − | { | ||
|- | |- | ||
| | | | ||
| − | = | + | :Subtract this subtractive 40 from the product of 10 by 10, which is 100; 60 remains. |
| − | + | :<math>\scriptstyle{\color{blue}{100-40=60}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והוצא אלו המ' הפוחתי' מכפל י' בי' שהוא ק' וישאר ס‫' |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Now, to complete the multiplication, it is left to multiply a subtractive 2 by a subtractive 2, which I say yields an additive 4. |
| − | + | :<math>\scriptstyle{\color{blue}{\left(-2\right)\sdot\left(-2\right)=4}}</math> | |
| − | + | |style="text-align:right;"|עתה חסר להשלים ההכפלה להכות <sup>ב'</sup> פוחתי' בב' פוחתי' אשר אומר שעושה ד' יותר | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | == | + | :When adding it to 60, it indeed yields 64 and this is the result of the multiplication of 10 minus 2 by 10 minus 2, that is 8 by 8. |
| − | + | :<math>\scriptstyle{\color{blue}{8\sdot8=\left(10-2\right)\sdot\left(10-2\right)=60+4=64}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אשר {{#annot:term|178,1206|TtZP}}בהוסיפו על{{#annotend:TtZP}} ס' עושה היטב ס"ד וככה עולה לכפול י' פחות ב' בי' פחות ב' דהיינו ח' בח‫' |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Therefore, if a subtractive multiplied by a subtractive would have yielded nothing, 4 should have been subtracted from 60, and this would have implied that 10 minus 2 multiplied by 10 minus 2, i.e. 8 by 8, yields 60 and this is wrong. |
| − | + | |style="text-align:right;"|ולכן אם פחות מוכה בפחות לא היה עושה כלום היה ראוי להוציא ד' או לקבצם מס‫'<br> | |
| − | + | א"כ ימשך כי י' פחות ב' מוכה בי' פחות ב' דהיינו ח' בח' יעשה ס' וזה יהיה כזב | |
| − | + | |- | |
| − | + | |colspan=2| | |
| − | + | :<math>\scriptstyle{\color{blue}{\left(-2\right)\sdot\left(-2\right)=0\longrightarrow8\sdot8=\left(10-2\right)\sdot\left(10-2\right)=60\longrightarrow impossible}}</math> | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | { | ||
|- | |- | ||
| | | | ||
| − | + | :And if a subtractive multiplied by a subtractive, meaning the subtractive 2 multiplied by the subtractive 2 would have yielded a subtractive 4, this 4 should have been subtracted from 60 and 56 would have remained, and this would have implied that 10 minus 2 multiplied by 10 minus 2, i.e. 8 by 8, yields 56 and this is also wrong. | |
| − | == | + | |style="text-align:right;"|ואם פחות מוכה בפחות רצוני אלו הב' פוחתים {{#annot:term|358,2025|vDc8}}מוכים ב{{#annotend:vDc8}}ב' פוחתי' יעשה ד' פוחתי‫'<br> |
| − | + | זה הד' יצטרך לגרעו מס' וישאר נ"ו<br> | |
| − | |style="text-align:right;"| | + | וימשך א"כ כי י' פחות ב' מוכי' בי' פחות ב' דהיינו ח' מוכה בח' יעשה נ"ו וזה ג"כ יהיה כזב |
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{\left(-2\right)\sdot\left(-2\right)=-4\longrightarrow8\sdot8=\left(10-2\right)\sdot\left(10-2\right)=60-4=56\longrightarrow impossible}}</math> | ||
| + | |- | ||
| + | |Hence, a subtractive multiplied by a subtractive is necessarily additive. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\left(-\right)\times\left(-\right)=\left(+\right)}}</math> | ||
| + | |style="text-align:right;"|א"כ פחות מוכה בפחות מחויב <sup>הוא</sup> שיעשה יותר | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :10 minus 2 by 10 minus 2 yields 64. |
| − | + | |style="text-align:right;"|י' פחות ב' בי' פחות ב' עולה ס"ד | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|} | |} | ||
{| | {| | ||
|- | |- | ||
| | | | ||
| − | |||
| − | |style="text-align:right;"| | + | ==== <span style=color:Green>Multiplication of a Number minus a Root by a Number minus a Root - when the numbers are identical and the roots are identical</span> ==== |
| + | | | ||
| + | |- | ||
| + | |If you wish to multiply a number minus a root by a number minus a root, when the numbers are identical and the roots are identical: | ||
| + | |style="width:45%;text-align:right;"|<big>ואם</big> רצית לכפול מספר פחות שרש במספר פחות שרש בהיות המספרי' שוים והשרשי' זה לזה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *{{#annot:(3-√5)×(3-√5)|737|H3RC}}Suppose you wish to multiply 3 minus a root of 5 by 3 minus a root of 5. |
| − | + | :<math>\scriptstyle\left(3-\sqrt{5}\right)\times\left(3-\sqrt{5}\right)</math> | |
| − | + | |style="text-align:right;"|ונניח שרצית לכפול ג' פחות שרש ה' בג' פחות שרש ה‫'{{#annotend:H3RC}} | |
| − | + | |- | |
| − | + | |You should multiply the numbers by each other: | |
| − | + | |style="text-align:right;"|הנך צריך להכות המספרי' זה על זה | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | = | + | :I.e. 3 by 3; the result is 9. |
| − | + | :<math>\scriptstyle{\color{blue}{3\sdot3=9}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|דהיינו ג' בג' ועולה ט‫' |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Add the number of one of the roots, which is 5, to this number, which is 9; they are 14. Keep it. |
| − | + | :<math>\scriptstyle{\color{blue}{9+5=14}}</math> | |
| − | + | |style="text-align:right;"|ועל מספר זה מט' תוסיף המספר מאחד השרשים שהוא ה' ויהיו י"ד ותשמרם | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | + | :Then, sum up the numbers, i.e. 3 and 3; they are 6. | |
| − | + | |style="text-align:right;"|אח"כ תקבץ המספרי' יחד דהיינו ג' וג' ויהיו ו‫' | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Convert 6 into a root; you get a root of 36. |
| − | + | |style="text-align:right;"|וזה הו' תביא אל שרש ויהיה לך שרש ל"ו | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | ויהיה לך | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | == | + | :Multiply a root of 36 by one of the roots, which is a subtractive 5; the result is a subtractive root of 180. |
| − | + | :<math>\scriptstyle{\color{blue}{\left(3+3\right)\sdot\left(-\sqrt{5}\right)=6\sdot\left(-\sqrt{5}\right)=\sqrt{36}\sdot\left(-\sqrt{5}\right)=-\sqrt{180}}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ותכפול שרש ל"ו באחד השרשים שהוא <sup>שרש</sup> ה' שהוא פחות ועולה שרש ק"פ פוחת |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Subtract the root of 180 from the number 14 you kept; 14 minus a root of 180 remains and this is the result of multiplication of 3 minus a root of 5 by 3 minus a root of 5. |
| − | + | :<math>\scriptstyle{\color{blue}{\left(3-\sqrt{5}\right)\times\left(3-\sqrt{5}\right)=14-\sqrt{180}}}</math> | |
| − | + | |style="text-align:right;"|ושרש ק"פ זה {{#annot:term|181,1232|o28u}}תוציא מ{{#annotend:o28u}}מספר י"ד אשר שמרת וישאר י"ד פחות שרש ק"פ<br> | |
| − | + | וככה עולה כפל ג' פחות שרש ה' בג' פחות שרש ה‫' | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |Also, if you want to multiply these or similar ones by another method of multiplying a number and a root by a number and a root - as I have shown you previously, you should do as follows: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<big>עוד</big> אם רצית לכפול כפל זה או דומה לו באופן אחר אשר הראיתיך לפנים לכפול מספר ושרש במספר ושרש ככה ראוי לך לעשות |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :First multiply the numbers by each other: |
| − | + | |style="text-align:right;"| {{#annot:term|185,1230|AGhb}}כפול{{#annotend:AGhb}} ראשונה המספרי' <sup>זה</sup> על זה | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | על | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :I.e. 3 by 3; the result is 9. |
| + | :<math>\scriptstyle{\color{blue}{3\sdot3=9}}</math> | ||
| + | |style="text-align:right;"|דהיינו ג' על ג' ועולה ט‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Then, multiply those that yield an additive, i.e, the subtractive root of 5 by the subtractive root of 5 that yield an additive root of 25; and this root is 5. |
| − | + | :<math>\scriptstyle{\color{blue}{\left(-\sqrt{5}\right)\sdot\left(-\sqrt{5}\right)=\sqrt{25}=5}}</math> | |
| − | + | |style="text-align:right;"|אח"כ תכה ‫<ref>4r</ref>עוד העושה יותר דהיינו שרש ה' הפוחת בשרש ה' הפוחת שהוא <s>ע</s> עושה שרש כ"ה יותר וזה השרש הוא ה‫' | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | אח"כ | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | = | + | :Sum the 5 with the product of the numbers, which is 9; the result is 14. Keep it. |
| − | + | :<math>\scriptstyle{\color{blue}{9+5=14}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וקבץ זה הה' עם {{#annot:term|241,1230|bonD}}כפל ה{{#annotend:bonD}}מספרי' שהיה ט' ויעלה י"ד ותשמרם |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Now, multiply 3 by the subtractive root of 5 crosswise; you get a subtractive root of 45 for each of the products. |
| − | + | :<math>\scriptstyle{\color{blue}{3\sdot\left(-\sqrt{5}\right)=-\sqrt{45}}}</math> | |
| − | + | |style="text-align:right;"|ועתה תכה ג' <sup>ב</sup>פחות שרש ה' בשתי וערב ויהיה לך שרש מ"ה לכל אחת מההכאות שהוא פוחת | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | = | + | :So, you have twice a subtractive root of 45. Therefore, multiply 2 by a root of 45; the result is a subtractive root of 180. |
| − | + | :<math>\scriptstyle{\color{blue}{2\sdot\left(-\sqrt{45}\right)=\sqrt{180}}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ולכן יהיה לך ב' פעמי' שרש מ"ה פוחת ולזה תכה ב' בשרש מ"ה ועולה שרש ק"פ פוחת |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Subtract the root of 180 from the 14 you kept; 14 minus a root of 180 remains and this is the result of multiplication of 3 minus a root of 5 by 3 minus a root of 5. |
| + | :<math>\scriptstyle{\color{blue}{\left(3-\sqrt{5}\right)\times\left(3-\sqrt{5}\right)=14-\sqrt{180}}}</math> | ||
| + | |style="text-align:right;"|ושרש ק"פ זה תוציא מי"ד אשר שמרת וישאר י"ד פחות שרש ק"פ וככה עולה הכאת ג' פחות שרש ה' בג' פחות שרש ה‫' | ||
|- | |- | ||
| − | | | + | |You can carry out this type of multiplication also by the aforementioned methods. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אשר הכאה זו תוכל ג"כ לעשותה באופני' האמורי' למעלה |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :3 minus a root of 5 by 3 minus a root of 5 yields 14 minus a root of 180. |
| − | + | |style="text-align:right;"|ג' פחות שרש ה' בג' פחות שרש ה' עושה י"ד פחות שרש ק"פ | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|} | |} | ||
{| | {| | ||
| Line 3,533: | Line 1,781: | ||
| | | | ||
| − | == | + | ==== <span style=color:Green>Multiplication of a Number and a Root by a Number minus a Root - when the numbers are identical and the roots are identical</span> ==== |
| − | + | | | |
| − | |||
|- | |- | ||
| − | | | + | |If you wish to multiply a number plus a root by a number minus a root: |
| − | |style="text-align:right;"| | + | |style="width:45%;text-align:right;"|<big>ואם</big> רצית לכפול מספר ושרש במספר פחות שרש |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *{{#annot:(5+√3)×(5-√3)|737|YkgW}}Suppose you wish to multiply 5 plus a root of 3 by 5 minus a root of 3. |
| − | + | :<math>\scriptstyle\left(5+\sqrt{3}\right)\times\left(5-\sqrt{3}\right)</math> | |
| − | + | |style="text-align:right;"|נניח שרצית לכפול ה' ושרש ג' בה' פחות שרש ג‫'{{#annotend:YkgW}} | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |You should multiply the numbers by each other: |
| − | + | |style="text-align:right;"|אתה צריך להכות המספרי' זה על זה | |
|- | |- | ||
| | | | ||
| − | + | :I.e. 5 by 5; the result is 25. Keep it. | |
| − | == | + | :<math>\scriptstyle{\color{blue}{5\sdot5=25}}</math> |
| − | + | |style="text-align:right;"|דהיינו ה' על ה' ועולה כ"ה ותשמרם | |
| − | |style="text-align:right;"| | + | |- |
| + | |Now, multiply the additive root by the number on the other side: | ||
| + | |style="text-align:right;"|עתה תכה השרש שהוא יותר במספר שהוא בצד האחר | ||
| + | |- | ||
| + | | | ||
| + | :I.e. a root of 3 by 5; the result is a root of 75. | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{3}\sdot5=\sqrt{75}}}</math> | ||
| + | |style="text-align:right;"|דהיינו שרש ג' בה' ועולה שרש ע"ה | ||
| + | |- | ||
| + | |Then, multiply the subtractive root by the number on the other side: | ||
| + | |style="text-align:right;"|‫[אח"כ תכה [השרש הפוחת] במספר [אשר בצד האחר‫] | ||
| + | |- | ||
| + | | | ||
| + | :I.e. a root of 3 by 5; the result is a subtractive root of 75. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(-\sqrt{3}\right)\sdot5=-\sqrt{75}}}</math> | ||
| + | |style="text-align:right;"|דהיינו [שרש ג' בה' ועולה] שרש מע"ה]‫<ref>marg.</ref> וזה השרש בא להיות פוחת | ||
| + | |- | ||
| + | |Since a subtractive multiplied by an additive yields a subtractive. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\left(-\right)\times\left(+\right)=\left(-\right)}}</math> | ||
| + | |style="text-align:right;"|בעבור כי פחות במוסיף עושה פחות | ||
| + | |- | ||
| + | |The subtractive should be subtracted from the additive root, but since they are equal - nothing remains. | ||
| + | |style="text-align:right;"|וזה {{#annot:term|789,2535|Xmmo}}הנפחת{{#annotend:Xmmo}} צריך להוציא מהשרש המוסיף ומפני היותם שוים ישאר לא כלום | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Therefore, what remains from the multiplication until now is only the product of the numbers by each other, i.e. 25 |
| − | + | |style="text-align:right;"|ולכן לא ישאר עד עתה מהכפל רק אשר היה מכפל המספרי' זה על זה דהיינו כ"ה | |
| − | + | |- | |
| − | + | |I remind you that whenever you multiply a number and a root that are the same as the number minus the root on the other term [<math>\scriptstyle{\color{OliveGreen}{\left(a+\sqrt{b}\right)\times\left(a-\sqrt{b}\right)}}</math>], you do not have to multiply the numbers by the roots, because the subtractive cancels the additive, as they are equal. | |
| − | + | |style="text-align:right;"|ולכן אזכירך שבכל פעם שיבואך הכאת מספר ושרש {{#annot:term|429,1247|1WQx}}שוים ל{{#annotend:1WQx}}מספר פוחת שרש בצד האחר אינך צריך להכות המספרי' בשרשי' <sup>בעבור</sup> כי הפוחת ממעיט המוסיף מפני השואתם | |
| − | + | |- | |
| − | + | |Therefore, the only thing left for you to do in [this type of] multiplication is to multiply the additive root by the subtractive root. | |
| − | + | :<math>\scriptstyle{\color{OliveGreen}{\sqrt{}\times\left(-\sqrt{}\right)}}</math> | |
| − | + | |style="text-align:right;"|ולכן אין צריך לך לעשות מהכפל עתה רק להכות השרש שהוא מוסיף בשרש שהוא פוחת | |
| − | + | |- | |
| − | + | | | |
| − | + | :I.e. the additive root of 3 by the subtractive root of 3 that yields a subtractive root of 9, which is 3. | |
| − | + | :<math>\scriptstyle{\color{blue}{\sqrt{3}\sdot\left(-\sqrt{3}\right)=-\sqrt{9}=-3}}</math> | |
| − | + | |style="text-align:right;"|דהיינו שרש ג' המוסיף בשרש ג' הפוחת העושה שרש ט' פוחת שהוא ג‫' | |
| − | + | |- | |
| − | שרש | + | | |
| + | :Subtract the 3 from 25; 22 remains, and this is the result of multiplication of 5 plus a root of 3 by 5 minus a root of 3. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(5+\sqrt{3}\right)\times\left(5-\sqrt{3}\right)=25-3=22}}</math> | ||
| + | |style="text-align:right;"|וזה הג' תוציא מכ"ה וישאר כ"ב<br> | ||
| + | וככה עולה הכאת ה' ושרש ג' בה' פחות שרש ג‫' | ||
| + | |- | ||
| + | | | ||
| + | :5 plus a root of 3 by 5 minus a root of 3 yields 22. | ||
| + | |style="text-align:right;"|ה' ושרש ג' בה' פחות שרש ג' עולה כ"ב | ||
|} | |} | ||
{| | {| | ||
|- | |- | ||
| | | | ||
| − | |||
| − | |style="text-align:right;"| | + | ==== <span style=color:Green>Multiplication of a Number and a Root by a Number minus a Root</span> ==== |
| + | |||
| + | | | ||
| + | |- | ||
| + | |I also want to show you another rule common to all [types of] multiplications of numbers and roots either equal or unequal, when one term is additive and the other term is subtractive: | ||
| + | |style="width:45%;text-align:right;"|<big>עוד</big> רצוני להראותך כלל אחר משותף לכל כפל שוה יהיה ממספר ושרש או לא יהיה שוה בהיות יותר מצד אחד ופחות מהאחר | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *{{#annot:(3+√4)×(5-√9)|737|zo1g}}Suppose you wish to multiply 3 plus a root of 4 by 5 minus a root of 9. |
| − | + | :<math>\scriptstyle\left(3+\sqrt{4}\right)\times\left(5-\sqrt{9}\right)</math> | |
| − | + | |style="text-align:right;"|נניח שרצית לכפול ג' ושרש ד' בה' פחות שרש ט‫'{{#annotend:zo1g}} | |
| − | + | |- | |
| − | + | |You should multiply the numbers by each other. | |
| − | + | |style="text-align:right;"|הנך צריך עתה להכות המספרי' זה על זה | |
| − | + | |- | |
| − | + | | | |
| − | + | :I.e. 3 by 5; the result is 15; keep it on one side. | |
| − | + | :<math>\scriptstyle{\color{blue}{3\sdot5=15}}</math> | |
| − | + | |style="text-align:right;"|דהיינו ג' בה' ועולה ט"ו ושמרם בצד אחד | |
| − | + | |- | |
| − | + | |Then, multiply by the number of the other term. | |
| − | + | |style="text-align:right;"|אח"כ תכה במספר מהצד האחר | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | = | + | :Meaning, a root of 4 by 5; the result is a root of 100. |
| − | + | :<math>\scriptstyle{\color{blue}{\sqrt{4}\sdot5=\sqrt{100}}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|רצוני שרש ד' בה' ועולה שרש ק‫' |
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Add it to the product of the numbers by each other, which is 15; you get 15 plus a root of 100. |
| − | + | :<math>\scriptstyle{\color{blue}{15+\sqrt{100}}}</math> | |
| − | + | |style="text-align:right;"|הוסיפהו על כפל המספרי' זה על זה שהוא ט"ו ויהיה לך ט"ו ושרש ק‫' | |
| − | + | |- | |
| − | + | |Now, multiply the subtractive root by the number of the other term: | |
| − | + | |style="text-align:right;"|עתה תכה השרש הפוחת במספר הצד האחר | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | == | + | :I.e. a subtractive root of 9 by 3; the result is a subtractive root of 81. |
| − | + | :<math>\scriptstyle{\color{blue}{\left(-\sqrt{9}\right)\sdot3=-\sqrt{81}}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|דהיינו שרש ט' הפוחת בג' ועולה שרש פ"א הפוחת |
| + | |- | ||
| + | |Keep the subtractive root to be subtracted from the sum we produced above. | ||
| + | |style="text-align:right;"|ותשמור להוציא זה השרש הפוחת מהסכום שעשינו למעלה | ||
| + | |- | ||
| + | |Then, multiply the subtractive root by the additive root of the other term: | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\left(-\sqrt{}\right)\times\sqrt{}}}</math> | ||
| + | |style="text-align:right;"|אח"כ תכה השרש הפוחת מצד אחד בשרש המוסיף מהצד האחר | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :Which is a subtractive root of 9 by an additive root of 4; you get a subtractive root of 36. |
| + | :<math>\scriptstyle{\color{blue}{\left(-\sqrt{9}\right)\sdot\sqrt{4}=-\sqrt{36}}}</math> | ||
| + | |style="text-align:right;"|שהוא שרש ט' הפוחת בשרש ד' המוסיף ויהיה לך שרש ל"ו פוחת | ||
| + | |- | ||
| + | |Subtract these two subtractive roots from the sum above. | ||
| + | |style="text-align:right;"|ואלו שני השרשי' הפוחתי' תוציא מהסכום אשר למעלה | ||
| + | |- | ||
| + | | | ||
| + | :15 plus a root of 100 minus a root of 81 minus a root of 36 remains. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(3+\sqrt{4}\right)\times\left(5-\sqrt{9}\right)=15+\sqrt{100}-\sqrt{81}-\sqrt{36}}}</math> | ||
| + | |style="text-align:right;"|וישאר ט"ו ושרש ק' פוחת שרש פ"א ופחות שרש ל"ו | ||
| + | |- | ||
| + | |The reason that we operate in this rule according to the way of the numbers whose roots are known is that we do it in order that the rule will be common to the known roots as well as the unknown roots. | ||
| + | |style="text-align:right;"|‫[והסבה]‫<ref>marg.</ref> בהיות כי אנחנו פעלנו בזה הכלל על דרך השרשי' ‫<ref>4v</ref>והמספרי' {{#annot:term|1073,2300|BaGE}}הנקובים להם שרשים'{{#annotend:BaGE}} ידועים הנה עשינו זה כדי שהכלל יהיה משותף קומונו בלעז לשרשי' ידועי' ולבלתי ידועי‫' | ||
| + | |- | ||
| + | | | ||
| + | :Because, when multiplying 3 plus a root of 4, which is 2, by 5 minus a root of 9, which is 3, 2 remains, we multiply 2 by 5; the result is ten. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(3+\sqrt{4}\right)\times\left(5-\sqrt{9}\right)=\left(3+2\right)\sdot\left(5-3\right)=5\sdot2=10}}</math> | ||
| + | |style="text-align:right;"|בהיות כי בהכות ג' ושרש ד' שהוא ב' בה' פחות שרש ט' שהוא ג' ישאר ב' ‫[ונכה ב' בה']‫<ref>marg.</ref> ועולה עשרה | ||
| + | |- | ||
| + | | | ||
| + | :This is also the result of the above multiplication that is: | ||
| + | |style="text-align:right;"|וג"כ ככה עולה ההכאה של מעלה שהיא | ||
| + | |- | ||
| + | | | ||
| + | :15 added to a root of 100, which is ten, yields 25. | ||
| + | |style="text-align:right;"|ט"ו {{#annot:term|178,2083|5gSG}}מחובר עם{{#annotend:5gSG}} שרש ק' שהוא עשרה ועושה כ"ה | ||
| + | |- | ||
| + | | | ||
| + | :When we subtract the two subtractive roots from 25 that are a root of 81, which is 9, and a root of 36, which is 6, that are 15, ten remains, which is this product and this is the result of 3 plus a root of 4 multiplied by 5 minus a root of 9. | ||
| + | |style="text-align:right;"|ו{{#annot:term|181,1232|vluk}}בהוציאנו מ{{#annotend:vluk}}זה שני השרשי' {{#annot:term|789,2031|CZ3Y}}הגורעי'{{#annotend:CZ3Y}} שהם שרש פ"א שהוא ט' ושרש ל"ו שהוא ו' שהם עולים ט"ו מאלו הכ"ה וישארו עשרה הכפל ההוא<br> | ||
| + | וככה עולה ג' ושרש ד' מוכה בה' פחות שרש ט‫' | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{\left(3+\sqrt{4}\right)\times\left(5-\sqrt{9}\right)=15+\sqrt{100}-\sqrt{81}-\sqrt{36}=15+10-9-6=25-15=10}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :3 plus a root of 4 by 5 minus a root of 9 yields ten. | ||
| + | |style="text-align:right;"|ג' ושרש ד' בה' פחות שרש ט' עולה עשרה | ||
| + | |} | ||
| + | {| | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | |
| − | + | ==== <span style=color:Green>Multiplication of a Root by a Root minus a Number</span> ==== | |
| − | + | | | |
| − | + | |- | |
| − | + | |Know also, if you wish to multiply a root by a root minus a number: | |
| − | + | |style="width:45%;text-align:right;"|<big>ודע</big> עוד אם רצית לכפול שרש בשרש פחות מספר | |
| − | + | |- | |
| − | + | | | |
| − | + | *{{#annot:√8×(√8-2)|737|p4H3}}Suppose you wish to multiply a root of 8 by a root of 8 minus 2. | |
| − | + | :<math>\scriptstyle\sqrt{8}\times\left(\sqrt{8}-2\right)</math> | |
| − | + | |style="text-align:right;"|נניח שרצית לכפול שרש ח' בשרש ח' פחות ב‫'{{#annotend:p4H3}} | |
| − | + | |- | |
| − | + | |You should multiply the roots by each other: | |
| − | + | |style="text-align:right;"|הנך צריך להכות השרשי' זה על זה | |
| − | + | |- | |
| − | + | | | |
| − | |} | + | :Meaning that you multiply the root of 8 by the root of 8; it yields 8. |
| − | + | :<math>\scriptstyle{\color{blue}{\sqrt{8}\sdot\sqrt{8}=8}}</math> | |
| − | == Appendix: Bibliography == | + | |style="text-align:right;"|רצוני שתכה שרש ח' בשרש ח' ועושה ח‫' |
| − | '''''Aliabraa Argibra''''' / by Maestro Dardi (Pisa, 14th century)<br> | + | |- |
| − | – Hebrew translation –<br> | + | |Since the roots are equal, it is the same as if you multiply one of them by itself, meaning that it yields the number by which the root is denominated [= the radicand]. |
| − | by Mordecai (Angelo) Finzi (Mantua, d. 1475)<br> | + | :<math>\scriptstyle{\color{OliveGreen}{\sqrt{a}\times\sqrt{a}=\sqrt{a^2}=a}}</math> |
| − | '''''Jīblī al- Mūqabāla'''<br> | + | |style="text-align:right;"|מפני שהשרשי' שוים ושוה הדבר כאלו כפלת אחד מהם בעצמו רצוני שראוי שיעשה המספר {{#annot:term|1073,2300|asPd}}הנקוב שהוא לו שרש{{#annotend:asPd}} |
| + | |- | ||
| + | |If the roots are not identical, we say that this product is the root of the product of the numbers, by which the roots are denominated [= the radicands], one by the other. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\sqrt{a}\times\sqrt{b}=\sqrt{a\sdot b}}}</math> | ||
| + | |style="text-align:right;"|ואם השרשי' לא יהיו שוים אנחנו נאמ' שהכפל יהיה שרש הכפל אשר יעשה מהכאת המספרי' הנקובי' להיות להם שרשי' זה בזה | ||
| + | |- | ||
| + | | | ||
| + | :So, we say that it yields a root of 64, which is the product of 8 by 8; keep the 8, which is a root of 64. | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{8}\sdot\sqrt{8}=\sqrt{64}=8}}</math> | ||
| + | |style="text-align:right;"|ולכן נאמ' אנחנו שיעשה שרש מס"ד שהוא מהכאת ח' בח' ושמור זה הח' שהוא שרש מס"ד | ||
| + | |- | ||
| + | |Then, multiply the subtractive number by the root of the first term: | ||
| + | |style="text-align:right;"|ואח"כ תכפול המספר הפוחת משרש החלק האחד | ||
| + | |- | ||
| + | | | ||
| + | :I.e. 2 by the root of the other term, which is a root of 8; it yields a subtractive root of 32. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(-2\right)\sdot\sqrt{8}=-\sqrt{32}}}</math> | ||
| + | |style="text-align:right;"|דהיינו ב' בשרש החלק האחר שהוא בשרש ח' ועושה שרש מל"ב הפוחת | ||
| + | |- | ||
| + | | | ||
| + | :Therefore, subtract the subtractive root of 32 from 8, or say: from a root of 64; you get 8 minus a root of 32. | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{8}\times\left(\sqrt{8}-2\right)=\sqrt{64}-\sqrt{32}=8-\sqrt{32}}}</math> | ||
| + | |style="text-align:right;"|ולכן תוציא שרש מל"ב הפוחת חוץ <s>מל</s> מח‫' או אמור משרש ס"ד ויהיה לך ח' פחות שרש ל"ב | ||
| + | |- | ||
| + | |If the roots are not identical, or if the product of the numbers, whose roots are multiplied by each other, has no root, you should convert them into a root minus a root. | ||
| + | |style="text-align:right;"|ואם השרשים לא היו שוים או כי בהכאת המספרי' אשר הם להם שרשי' [זה על זה]‫<ref>marg.</ref> לא יהיה לה שרש אתה צריך להשיב שרש פחות שרש | ||
| + | |- | ||
| + | | | ||
| + | :As if you say: a root of 64 minus a root of 32 and this is the product of a root of 8 by a root of 8 minus 2. | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{8}\times\left(\sqrt{8}-2\right)=\sqrt{64}-\sqrt{32}=8-\sqrt{32}}}</math> | ||
| + | |style="text-align:right;"|כאלו תאמר שרש ס"ד פחות שרש ל"ב וככה עולה הכאת שרש ח' בשרש ח' פחות ב‫' | ||
| + | |- | ||
| + | | | ||
| + | :A root of 8 multiplied by a root of 8 minus 2 yields 8 minus a root of 32. | ||
| + | |style="text-align:right;"|שרש ח' מוכה בשרש ח' פחות ב' עולה ח' פחות שרש ל"ב | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== <span style=color:Green>Multiplication of a Root minus a Number by a Root minus a Number</span> ==== | ||
| + | | | ||
| + | |- | ||
| + | |If you wish to multiply a root minus a number by a root minus a number: | ||
| + | |style="width:45%;text-align:right;"|<big>ואם</big> רצית לכפול שרש פחות מספר בשרש פחות מספר | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:(√8-2)×(√10-3)|737|hEei}}Suppose you wish to multiply a root of 8 minus 2 by a root of ten minus 3. | ||
| + | :<math>\scriptstyle\left(\sqrt{8}-2\right)\times\left(\sqrt{10}-3\right)</math> | ||
| + | |style="text-align:right;"|ונניח שרצית לכפול שרש ח' פחות ב' בשרש עשרה פחות ג‫'{{#annotend:hEei}} | ||
| + | |- | ||
| + | |You should multiply the roots by each other: | ||
| + | |style="text-align:right;"|אתה צריך לכפול השרשי' זה על זה | ||
| + | |- | ||
| + | | | ||
| + | :Meaning a root of 8 by a root of ten; the result is a root of 80. Keep it. | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{8}\sdot\sqrt{10}=\sqrt{80}}}</math> | ||
| + | |style="text-align:right;"|רצוני שרש ח' בשרש עשרה ועולה שרש פ' ושמרהו | ||
| + | |- | ||
| + | |Then, multiply the subtractive numbers of each term: | ||
| + | |style="text-align:right;"|אח"כ תכפול המספרי' הפוחתי' מכל אחד מהחלקי‫' | ||
| + | |- | ||
| + | | | ||
| + | :They are a subtractive 2 by a subtractive 3; they yield an additive 6. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(-2\right)\sdot\left(-3\right)=6}}</math> | ||
| + | |style="text-align:right;"|שהם ב' פוחתי' בג' פוחתי' ועושים ו' מוספי‫' | ||
| + | |- | ||
| + | | | ||
| + | :Add the 6 to the root of 80; you have a root of 80 plus 6. | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{80}+6}}</math> | ||
| + | |style="text-align:right;"|תוסיף זה הו' על שרש פ' אשר שמרת ויהיה לך שרש פ' וו' יותר | ||
| + | |- | ||
| + | |Then, multiply the subtractive numbers by the counter roots crosswise: | ||
| + | |style="text-align:right;"|אח"כ תכפול המספרים שהם גורעי' בשרשי' שהם מנגדי' שתי וערב | ||
| + | |- | ||
| + | | | ||
| + | :I.e. the subtractive 2 on one side by the root on the other side; the result is a subtractive root of 40. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(-2\right)\sdot\sqrt{10}=-\sqrt{40}}}</math> | ||
| + | |style="text-align:right;"|דהיינו ב' הפוחתי' מהצד האחד בשרש אשר בצד האחר ועולה שרש מ' הפוחת | ||
| + | |- | ||
| + | | | ||
| + | :Multiply the other subtractive number, which is 3, [by] a root of 8 on the other side; you get a subtractive root of 72. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(-3\right)\sdot\sqrt{8}=-\sqrt{72}}}</math> | ||
| + | |style="text-align:right;"|אח"כ תכה המספר האחר הפוחת שהוא ג' פחות שרש ח' אשר הוא מהצד האחר ויהיה לך שרש ע"ב הפוחת | ||
| + | |- | ||
| + | | | ||
| + | :Now, subtract the subtractive roots that are a root of 40 and a root of 72 from the sum above; 6 plus a root of 80 minus a root of 40 minus a root of 72 remains and this is the result of multiplication of a root of 8 minus 2 by a root of ten minus 3. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(\sqrt{8}-2\right)\times\left(\sqrt{10}-3\right)=6+\sqrt{80}-\sqrt{40}-\sqrt{72}}}</math> | ||
| + | |style="text-align:right;"|עתה תוציא שני אלו השרשי' הפוחתי' מהסכום של מעלה שהוא שרש מ' ושרש ע"ב וישאר ו' ושרש פ' פחות שרש מ' ופחות שרש ע"ב<br> | ||
| + | וככה עולה כפל שרש ח' פחות ב' בשרש עשרה פחות ג‫' | ||
| + | |- | ||
| + | |Know that starting by multiplying the subtractive numbers first is the same as starting by multiplying the roots first. | ||
| + | |style="text-align:right;"|<big>ודע</big> כי הדבר שוה בהתחיל לכפול ראשונה <sup>מן</sup> המספרי' הגורעי' כמו בהתחיל ‫<ref>5r</ref>לכפול ראשונה מהשרשי‫' | ||
| + | |- | ||
| + | |Always remember to multiply first the multiplicands whose products are additive in order to sum up all that should be added in the product, then to subtract all that should be subtracted, as you did in the multiplication above. | ||
| + | |style="text-align:right;"|ותזכור לעולם לכפול ראשונה חלקי הכפל אשר מוכפלים יעשו יותר בסבת {{#annot:term|178,1165|tJmU}}חבר<s>ם</s> יחד{{#annotend:tJmU}} כל אשר מהכפל ראוי להוסיף להוציא אח"כ בסדר מה שראוי לגרוע כפי אשר עשית בכפל האמור | ||
| + | |- | ||
| + | | | ||
| + | :A root of 8 minus 2 by a root of 10 minus 3 yields 6 plus a root of [80] minus a root of 40 minus a root of 72. | ||
| + | |style="text-align:right;"|שרש ח' פחות ב' בשרש י' פחות ג' עולה ו' ושרש ח' גורע שרש מ' ופחות שרש ע"ב | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== <span style=color:Green>Multiplication of a Root minus a Number by a Root minus a Number - when the numbers are identical [and the roots are identical]</span> ==== | ||
| + | | | ||
| + | |- | ||
| + | |If you wish to multiply a root minus a number by a root minus a number, when the numbers are identical [and the roots are identical]: | ||
| + | |style="width:45%;text-align:right;"|<big>עוד</big> אם בקשת לכפול שרש פחות מספר בשרש פחות מספר בהיות המספרי' שוים זה לזה | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:(√12-2)×(√12-2)|737|zWp7}}Suppose you wish to multiply a root of 12 minus 2 by a root of 12 minus 2. | ||
| + | :<math>\scriptstyle\left(\sqrt{12}-2\right)\times\left(\sqrt{12}-2\right)</math> | ||
| + | |style="text-align:right;"|ונניח שרצית לכפול שרש י"ב פחות ב' בשרש י"ב פחות ב‫'{{#annotend:zWp7}} | ||
| + | |- | ||
| + | |First you should multiply the roots by each other: | ||
| + | |style="text-align:right;"|אתה צריך להכות ראשונה השרשי' זה בזה | ||
| + | |- | ||
| + | | | ||
| + | :I.e. a root of 12 by a root of 12; the result is 12. | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{12}\sdot\sqrt{12}=12}}</math> | ||
| + | |style="text-align:right;"|דהיינו שרש י"ב בשרש י"ב ועולה י"ב | ||
| + | |- | ||
| + | |Then, multiply the subtractive numbers by each other: | ||
| + | |style="text-align:right;"|ואח"כ תכפול המספרי' הפוחתי' זה על זה | ||
| + | |- | ||
| + | | | ||
| + | :I.e. a subtractive 2 by a subtractive 2; it yields an additive 4. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(-2\right)\sdot\left(-2\right)=4}}</math> | ||
| + | |style="text-align:right;"|דהיינו ב' פוחתי' בב' פוחתי' ועושי' ד' יותר | ||
| + | |- | ||
| + | | | ||
| + | :Add it to the 12; you get 16. Keep it. | ||
| + | :<math>\scriptstyle{\color{blue}{12+4=16}}</math> | ||
| + | |style="text-align:right;"|ותקבץ עם י"ב ויהיה לך י"ו ושמרם | ||
| + | |- | ||
| + | |Then, sum up the numbers together: | ||
| + | |style="text-align:right;"|ואח"כ קבץ המספרי' יחד | ||
| + | |- | ||
| + | | | ||
| + | :I.e. a subtractive 2 with a subtractive 2; you get a subtractive 4. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(-2\right)+\left(-2\right)=-4}}</math> | ||
| + | |style="text-align:right;"|דהיינו ב' פוחתים עם ב' פוחתי' ויהיו לך ד' פוחתי‫' | ||
| + | |- | ||
| + | | | ||
| + | :Multiply the 4 by one of the roots, meaning by a root of 12; you have a subtractive root of 192. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(-4\right)\sdot\sqrt{12}=-\sqrt{192}}}</math> | ||
| + | |style="text-align:right;"|וזה הד' תכפול באחד מהשרשי' רצוני בשרש י"ב ויהיה לך שרש מקצ"ב פוחת | ||
| + | |- | ||
| + | | | ||
| + | :Subtract this root from 16; 16 minus a root of 192 remains and this is the result of multiplication of a root of 12 minus 2 by a root of 12 minus 2. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(\sqrt{12}-2\right)\times\left(\sqrt{12}-2\right)=16-\sqrt{192}}}</math> | ||
| + | |style="text-align:right;"|וזה השרש תוציא מי"ו וישאר י"ו פחות שרש מקצ"ב וככה עולה כפל שרש י"ב פחות ב' בשרש י"ב פחות ב‫' | ||
| + | |- | ||
| + | |We can perform this multiplication by another method: | ||
| + | |style="text-align:right;"|<big>עוד</big> נוכל לעשות זה הכפל באופן אחר | ||
| + | |- | ||
| + | | | ||
| + | :After you multiply a root of 12 by a root of 12; which yields 12. | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{12}\sdot\sqrt{12}=12}}</math> | ||
| + | |style="text-align:right;"|אחר ש{{#annot:term|185,1230|f2dm}}כפלת{{#annotend:f2dm}} שרש י"ב בשרש י"ב שעושה י"ב | ||
| + | |- | ||
| + | | | ||
| + | :Then, a subtractive 2 by a subtractive 2; which yields an additive 4. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(-2\right)\sdot\left(-2\right)=4}}</math> | ||
| + | |style="text-align:right;"|ואח"כ ב' פוחתי' בב' פוחתי' שעושה ד' יותר | ||
| + | |- | ||
| + | | | ||
| + | :Summed together, it yields 16. | ||
| + | :<math>\scriptstyle{\color{blue}{12+4=16}}</math> | ||
| + | |style="text-align:right;"|ומקובצי' יחד עושה י"ו | ||
| + | |- | ||
| + | |You then multiply each root by the counter subtractive number crosswise: | ||
| + | |style="text-align:right;"|ואחר תכפול כל שרש עם המספר המקביל לו הפוחת בשתי וערב | ||
| + | |- | ||
| + | | | ||
| + | :Meaning a root of 12 by a subtractive 2; it yields a subtractive root of 48. | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{12}\sdot\left(-2\right)=-\sqrt{48}}}</math> | ||
| + | |style="text-align:right;"|רצוני שרש י"ב בב' הפוחת ועושה שרש מ"ח פוחת | ||
| + | |- | ||
| + | | | ||
| + | :Then, the other root of 12 by the other subtractive 2 on the other side; it yields a subtractive root of 48. | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{12}\sdot\left(-2\right)=-\sqrt{48}}}</math> | ||
| + | |style="text-align:right;"|ואח"כ השרש האחר מי"ב בב' האחר הפוחת אשר מצד האחר ועולה שרש ממ"ח פוחת | ||
| + | |- | ||
| + | | | ||
| + | :The two subtractive roots of 48 together are a subtractive root of 192. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(-\sqrt{48}\right)+\left(-\sqrt{48}\right)=2\sdot\left(-\sqrt{48}\right)=-\sqrt{192}}}</math> | ||
| + | |style="text-align:right;"|אשר יהיה בין שני כפלי שרשי מ"ח פוחתי' שרש מקצ"ב פוחתי‫' | ||
| + | |- | ||
| + | |You have this [type] of multiplication performed by the two methods. | ||
| + | |style="text-align:right;"|ויהיה לך הכפל הזה עשוי בשני ה{{#annot:term|469|0aqc}}אופני‫'{{#annotend:0aqc}} | ||
| + | |- | ||
| + | | | ||
| + | :It is 16 minus a root of 192. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(\sqrt{12}-2\right)\times\left(\sqrt{12}-2\right)=16-\sqrt{192}}}</math> | ||
| + | |style="text-align:right;"|והוא י"ו פחות שרש קצ"ב | ||
| + | |- | ||
| + | | | ||
| + | :A root of 12 minus 2 by a root of 12 minus 2 yields 16 minus a root of 192. | ||
| + | |style="text-align:right;"|שרש י"ב פחות ב' בשרש י"ב פחות ב' עולה י"ו פחות שרש קצ"ב | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== <span style=color:Green>Multiplication of a Root minus a Number by a Root and a Number</span> ==== | ||
| + | | | ||
| + | |- | ||
| + | |If you wish to multiply a root minus a number by a root plus a number: | ||
| + | |style="width:45%;text-align:right;"|<big>עוד</big> אם רצית לכפול שרש פחות מספר בשרש ומספר | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:(√15-3)×(√12+2)|737|ITr2}}Suppose you wish to multiply a root of 15 minus 3 by a root of 12 plus 2. | ||
| + | :<math>\scriptstyle\left(\sqrt{15}-3\right)\times\left(\sqrt{12}+2\right)</math> | ||
| + | |style="text-align:right;"|נניח שרצית לכפול שרש ט"ו פחות ג' בשרש י"ב וב‫'{{#annotend:ITr2}} | ||
| + | |- | ||
| + | |You should multiply the roots by each other: | ||
| + | |style="text-align:right;"|עתה צריך אתה לכפול השרשי' זה בזה | ||
| + | |- | ||
| + | | | ||
| + | :I.e. a root of 15 by a root of 12; the result is a root of 180. Keep it. | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{15}\sdot\sqrt{12}=\sqrt{180}}}</math> | ||
| + | |style="text-align:right;"|דהיינו שרש ט"ו בשרש י"ב ועולה שרש ק"פ ושמרהו | ||
| + | |- | ||
| + | |Now, multiply the additive number on one side by the root on the other side: | ||
| + | |style="text-align:right;"|עתה תכפול המספר היותר מהצד האחד בשרש אשר בצד האחר | ||
| + | |- | ||
| + | | | ||
| + | :I.e. a root of 2 by a root of 15; the result is a root of 60. | ||
| + | :<math>\scriptstyle{\color{blue}{2\sdot\sqrt{15}=\sqrt{60}}}</math> | ||
| + | |style="text-align:right;"|דהיינו ב' בשרש ט"ו ועולה שרש מס‫' | ||
| + | |- | ||
| + | | | ||
| + | :Add it to the root of 180 you kept; you have a root of 180 plus a root of 60. Keep them. | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{180}+\sqrt{60}}}</math> | ||
| + | |style="text-align:right;"|ו{{#annot:term|178,1210|6iBe}}קבצהו עם{{#annotend:6iBe}} שרש ק"פ אשר שמרת ויהיה לך שרש ק"פ ושרש ס' ושמרם | ||
| + | |- | ||
| + | |Then, multiply the subtractive number on one side by the root on the other side: | ||
| + | |style="text-align:right;"|אח"כ תכפול המספ' הפוחת מצד אחד בשרש אשר מצד אחר | ||
| + | |- | ||
| + | | | ||
| + | :I.e. the subtractive 3 by a root of 12; the result is a subtractive root of 108. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(-3\right)\sdot\sqrt{12}=-\sqrt{108}}}</math> | ||
| + | |style="text-align:right;"|דהיינו ג' פוחתי' בשרש י"ב ועולה שרש מק"ח הפוחת | ||
| + | |- | ||
| + | |Multiply also the subtractive number and the additive [number] by each other: | ||
| + | |style="text-align:right;"|ועוד תכפול המספרי' הפוחתי' והיותר מהשרשי' האחד באחר | ||
| + | |- | ||
| + | | | ||
| + | :I.e. the subtractive 3 by the additive 2; the result is a subtractive 6. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(-3\right)\sdot2=-6}}</math> | ||
| + | |style="text-align:right;"|דהיינו ג' הפוחתי' בב' המוסיפי' ועולי' ו' פוחתי‫' | ||
| + | |- | ||
| + | | | ||
| + | :Now, subtract the two subtractive products that are a subtractive root of 108 and a subtractive 6 from the mentioned sum, which is a root of 180 plus a root of 60; a root of 180 plus a root of 60 minus a root of 108 minus 6 remains. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(\sqrt{15}-3\right)\times\left(\sqrt{12}+2\right)=\sqrt{180}+\sqrt{60}-\sqrt{108}-6}}</math> | ||
| + | |style="text-align:right;"|עתה הוצא אלו שתי ה{{#annot:term|241,1229|lSOt}}הכפלות{{#annotend:lSOt}} הפוחתים שהם שרש מק"ח הפוחת וו' הפוחתי' מהסכום הנז' שהוא שרש מק"פ ושרש מס' וישאר שרש מק"פ ושרש מס' פחות שרש מק"ח פחות ו‫' | ||
| + | |- | ||
| + | |Always remember to multiply first the multiplicands whose products are additive, then multiply those [whose products are] subtractive, in order to subtract them from the additive. | ||
| + | |style="text-align:right;"|וזכור תמיד לכפול ‫<ref>5v</ref>ראשונה כל החלקי' מההכפלו' אשר {{#annot:term|358,2136|fHCE}}בהכפלם{{#annotend:fHCE}} יעשו {{#annot:term|788,2536|SWhc}}יותר{{#annotend:SWhc}} ואח"כ תכפול העושים פחות להוציאם מהרב | ||
| + | |- | ||
| + | | | ||
| + | :When multiplying a root of 15 minus 3 by a root of 12 plus 2, the result is a root of 180 plus a root of 60 minus a root of 108 minus the number 6. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(\sqrt{15}-3\right)\times\left(\sqrt{12}+2\right)=\sqrt{180}+\sqrt{60}-\sqrt{108}-6}}</math> | ||
| + | |style="text-align:right;"|והנה {{#annot:term|185,1230|eY2X}}בכפול{{#annotend:eY2X}} שרש ט"ו פחות <s>ב'</s> ג' בשרש י"ב וב' עולה שרש ק"פ ושרש ס' פחות שרש <s>ק"פ</s> ק"ח ופחות ו' מספרים | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== <span style=color:Green>Multiplication of a Root and a Number by a Root minus a Number - when the numbers are identical and the roots are identical</span> ==== | ||
| + | | | ||
| + | |- | ||
| + | |If you wish to multiply a root plus a number by a root minus a number, when the numbers are identical and the roots are identical. | ||
| + | |style="width:45%;text-align:right;"|<big>עוד</big> אם רצית לכפול שרש ומספר בשרש פחות מספר בהיות המספרי' שוים זה לזה והשרשי' זה לזה | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:(√8+2)×(√8-2)|737|E8mu}}Suppose you wish to multiply a root of 8 plus 2 by a root of 8 minus 2. | ||
| + | :<math>\scriptstyle\left(\sqrt{8}+2\right)\times\left(\sqrt{8}-2\right)</math> | ||
| + | |style="text-align:right;"|ונניח <s>ל</s> שרצית לכפול שרש ח' וב' <s>פחות</s> <sup>ב</sup>שרש ח' פחות ב‫'{{#annotend:E8mu}} | ||
| + | |- | ||
| + | |First you should multiply the roots by each other: | ||
| + | |style="text-align:right;"|הנך צריך ראשונה לכפול השרשי' זה בזה | ||
| + | |- | ||
| + | | | ||
| + | :I.e. a root of 8 by a root of 8; the result is a root of 8. Keep it. | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{8}\sdot\sqrt{8}=8}}</math> | ||
| + | |style="text-align:right;"|דהיינו שרש ח' בשרש ח' ועולה ח' ושמרם | ||
| + | |- | ||
| + | |Then, we continue to multiply the root on one side by the subtractive number on the other side. | ||
| + | |style="text-align:right;"|אח"כ נמשיך לכפול השרשי' אשר בצד אחד במספר אשר <sup>פוחת</sup> בצד האחר | ||
| + | |- | ||
| + | |Afterwards the additive number on one side by the root on the other side. | ||
| + | |style="text-align:right;"|ואח"כ המספר אשר בצד אחד שהוא יותר בשרש אשר בצד האחר | ||
| + | |- | ||
| + | |Since these two products are equal to one another, but one is additive and the other is subtractive, when they are summed together it yields nothing. | ||
| + | |style="text-align:right;"|ובעבור שתי אלה ההכפלות שהם {{#annot:term|461,1247|pUat}}שוות זו לזו{{#annotend:pUat}} עושה יותר והאחרת עושה פחות בחברם יחד עושה לא כלום | ||
| + | |- | ||
| + | |Therefore nothing should be done in this multiplication and its similar, except for multiplying the subtractive number by the additive number. | ||
| + | |style="text-align:right;"|ולכן לא יצטרך בכפל הזה <s>ובכר</s> ובכדומה לו לעשות דבר אחר רק לכפול המספרי' הפוחתי' במספרי' המוסיפי‫' | ||
| + | |- | ||
| + | | | ||
| + | :I.e. a subtractive 2 by an additive 2 that yields a subtractive 4. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(-2\right)\sdot\left(-2\right)=4}}</math> | ||
| + | |style="text-align:right;"|דהיינו בזה ב' פחות ב' בב' יותר שהוא עושה ד' פוחתי‫' | ||
| + | |- | ||
| + | | | ||
| + | :Subtract it from the product of the roots by each other, which is 8; 4 remains and this is the result of multiplication of a root of 8 plus 2 by a root of 8 minus 2, meaning the result is 4. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(\sqrt{8}+2\right)\times\left(\sqrt{8}-2\right)=8-4=4}}</math> | ||
| + | |style="text-align:right;"|והוצא מכפל השרשי' זה בזה שהוא ח' וישאר ד' וככה עולה כפל שרש ח' וב' בשרש ח' פחות ב' רצוני שעולה ד‫' | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== <span style=color:Green>Multiplication of a Root by a Root and a Root</span> ==== | ||
| + | | | ||
| + | |- | ||
| + | |If you wish to multiply a root by a root plus a root. | ||
| + | |style="width:45%;text-align:right;"|<big>ואם</big> רצית לכפול שרש בשרש ושרש | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:√5×(√7+√10)|737|DUGD}}Suppose you wish to multiply a root of 5 by a root of 7 plus a root of ten. | ||
| + | :<math>\scriptstyle\sqrt{5}\times\left(\sqrt{7}+\sqrt{10}\right)</math> | ||
| + | |style="text-align:right;"|ונניח שרצית לכפול שרש ה' בשרש ז' ושרש עשרה{{#annotend:DUGD}} | ||
| + | |- | ||
| + | |You should multiply the single root by each of the two other roots, or likewise if there were more, in the way we multiply a root by a root. | ||
| + | |style="text-align:right;"|הנך צריך לכפול השרש היחיד באחד אחד מאותם השנים באופן אשר נכפול שרש בשרש וכן אם היו יותר בזה האופן | ||
| + | |- | ||
| + | | | ||
| + | :Multiply first a root of 5 by a root of 7; the result is a root of 35. Keep it. | ||
| + | ::<math>\scriptstyle{\color{blue}{\sqrt{5}\sdot\sqrt{7}=\sqrt{35}}}</math> | ||
| + | |style="text-align:right;"|תכפול ראשונה שרש ה' בשרש ז' שעולה שרש ל"ה ושמרהו | ||
| + | |- | ||
| + | | | ||
| + | :Then, multiply also a root of 5 by a root of ten; the result is a root of 50. | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{5}\sdot\sqrt{10}=\sqrt{50}}}</math> | ||
| + | |style="text-align:right;"|אח"כ תכפול עוד שרש ה' בשרש עשרה ועולה שרש נ‫' | ||
| + | |- | ||
| + | | | ||
| + | :Sum them together; you get a root of 35 plus a root of 50 and this is the result of multiplication of a root of 5 by a root of 7 plus a root of ten. | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{5}\times\left(\sqrt{7}+\sqrt{10}\right)=\sqrt{35}+\sqrt{50}}}</math> | ||
| + | |style="text-align:right;"|וקבצם יחד ויהיה לך שרש ל"ה ושרש נ‫'<br> | ||
| + | וככה עולה הכאת שרש ה' בשרש ז' ושרש עשרה | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== <span style=color:Green>Multiplication of a Root by a Root minus a Root</span> ==== | ||
| + | | | ||
| + | |- | ||
| + | |If you wish to multiply a root by a root minus a root. | ||
| + | |style="width:45%;text-align:right;"|<big>ואם</big> רצית להכות שרש בשרש פחות שרש | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:√5×(√12-√8)|737|PUFC}}Suppose you wish to multiply a root of 5 by a root of 12 minus a root of 8. | ||
| + | :<math>\scriptstyle\sqrt{5}\times\left(\sqrt{12}-\sqrt{8}\right)</math> | ||
| + | |style="text-align:right;"|ונניח שבקשת לכפול שרש ה' בשרש י"ב פחות שרש ח‫'{{#annotend:PUFC}} | ||
| + | |- | ||
| + | | | ||
| + | :You should first multiply a root of 5 by a root of 12; the result is a root of 60. Keep it. | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{5}\sdot\sqrt{12}=\sqrt{60}}}</math> | ||
| + | |style="text-align:right;"|הנך צריך לכפול ראשונה שרש ה' בשרש י"ב שעולה שרש ס' ושמרם | ||
| + | |- | ||
| + | | | ||
| + | :Then, multiply a root of 5 by the subtractive root, which is a root of 8; the result is a subtractive root of 40. | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{5}\sdot\left(-\sqrt{8}\right)=-\sqrt{40}}}</math> | ||
| + | |style="text-align:right;"|אח"כ תכפול שרש ה' בשרש הפוחת שהוא שרש ח' ועולה שרש מ' פוחת | ||
| + | |- | ||
| + | | | ||
| + | :Now, subtract the subtractive product from the product you kept; a root of 60 minus a root of 40 remains and this is the result of multiplication of a root of 5 by a root of 12 minus a root of 8. | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{5}\times\left(\sqrt{12}-\sqrt{8}\right)=\sqrt{60}-\sqrt{40}}}</math> | ||
| + | |style="text-align:right;"|ועתה {{#annot:term|181,1232|WwoT}}הוצא ה{{#annotend:WwoT}}כפל הזה {{#annot:term|789,2031|vkgW}}הגורע{{#annotend:vkgW}} מהכפל אשר שמרת וישאר שרש ס' פחות שרש מ' וככה עולה הכאת שרש ה' בשרש י"ב פחות שרש ח‫' | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== <span style=color:Green>Multiplication of Two Roots by Two other Roots</span> ==== | ||
| + | | | ||
| + | |- | ||
| + | |Likewise, if you wish to multiply two roots by two other roots. | ||
| + | |style="width:45%;text-align:right;"|<big>עוד</big> אם רצית לכפול ב' שרשים בב' שרשים אחרים | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:(√5+√7)×(√10+√15)|737|r71h}}Suppose you wish to multiply a root of 5 plus a root of 7 by a root of ten plus a root of 15. | ||
| + | :<math>\scriptstyle\left(\sqrt{5}+\sqrt{7}\right)\times\left(\sqrt{10}+\sqrt{15}\right)</math> | ||
| + | |style="text-align:right;"|ונניח שבקש<sup>ת</sup> לכפול שרש ה' ושרש ז' בשרש עשרה ושרש ט"ו{{#annotend:r71h}} | ||
| + | |- | ||
| + | |Multiply these roots in the aforementioned way of multiplying numbers and roots: | ||
| + | |style="text-align:right;"|תכפול אלו השרשי' ב{{#annot:term|469|LfEg}}אופן{{#annotend:LfEg}} האמור בהכאת מספרי' ושרשי‫' | ||
| + | |- | ||
| + | |First, start to multiply the first counter roots: | ||
| + | |style="text-align:right;"|וראשונה תתחיל לכפול מהשרשי' הראשוני' המתנגדי‫' | ||
| + | |- | ||
| + | | | ||
| + | :I.e. a root of 5 by a root of ten; the result is a root of 50. Keep it. | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{5}\sdot\sqrt{10}=\sqrt{50}}}</math> | ||
| + | |style="text-align:right;"|דהיינו שרש ה' בשרש עשרה ועולה שרש נ' ושמרם | ||
| + | |- | ||
| + | |Multiply the first counter roots by the other roots crosswise: | ||
| + | |style="text-align:right;"|אח"כ {{#annot:term|2521,2522|vvgf}}תכפול בשתי וערב{{#annotend:vvgf}} השרשי' הראשוני' המתנגדי' בשרשי' השניים | ||
| + | |- | ||
| + | | | ||
| + | :I.e. a root of 5 by a root of 15; the result is a root of 75. | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{5}\sdot\sqrt{15}=\sqrt{75}}}</math> | ||
| + | |style="text-align:right;"|דהיינו שרש ה' בשרש ט"ו ועולה שרש ע"ה | ||
| + | |- | ||
| + | | | ||
| + | :Then, multiply a root of ten by a root of 7; the result is a root of 70. | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{10}\sdot\sqrt{7}=\sqrt{70}}}</math> | ||
| + | |style="text-align:right;"|אח"כ תכה שרש עשרה בשרש ז' ועולה שרש ע‫' | ||
| + | |- | ||
| + | | | ||
| + | :Sum up these two products with the first; you get a root of 50 [plus a root of 75] and a root of 70. | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{50}+{\color{red}{\sqrt{75}}}+\sqrt{70}}}</math> | ||
| + | |style="text-align:right;"|וקבץ שתי אלו ההכאות עם הראשונה ויהיו לך שרש נ' ושרש ע‫' | ||
| + | |- | ||
| + | |Then, multiply the two last counter roots by each other: | ||
| + | |style="text-align:right;"|אח"כ תכפול שני השרשי' האחרוני' המתנגדי' זה על זה | ||
| + | |- | ||
| + | | | ||
| + | :I.e. a root of 7 by a root of 15; the result is a root of 105. | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{7}\sdot\sqrt{15}=\sqrt{105}}}</math> | ||
| + | |style="text-align:right;"|דהיינו ‫<ref>6r</ref>שרש ז' בשרש ט"ו ועולה שרש ק"ה | ||
| + | |- | ||
| + | | | ||
| + | :Sum it with the products of the three other roots; you get a sum of a root of 50, a root of 75, a root of 70 and a root of 105; and this is the result of multiplication of a root of 5 plus a root of 7 by a root of ten plus a root of 15. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(\sqrt{5}+\sqrt{7}\right)\times\left(\sqrt{10}+\sqrt{15}\right)=\sqrt{50}+\sqrt{75}+\sqrt{70}+\sqrt{105}}}</math> | ||
| + | |style="text-align:right;"|וקבצם עם הכאות שלשת השרשי' האחרים והיה לך שרש נ' ושרש ע"ה ושרש ע' ושרש ק"ה כלם יחד וככה עושה להכות שרש ה' ושרש ז' בשרש עשרה ושרש ט"ו | ||
| + | |- | ||
| + | |If you wish to start multiplying from the later roots apply the way of multiplying numbers crosswise in boxes casillas in foreign language. | ||
| + | |style="text-align:right;"|<big>ואם</big> בקשת להתחיל לכפול מן השרשי' האחרוני' תהיה רודף אופן כפל [המספרים בדרך]‫<ref>marg.</ref> הבתי' קאסילי בלעז בשתי וערב | ||
| + | |- | ||
| + | |You can write the multiplication of the roots in the way the numbers are written. | ||
| + | |style="text-align:right;"|וכן תוכל לכתוב כפל השרשי' באופן שבאי' לכתוב המספרים | ||
| + | |- | ||
| + | | | ||
| + | :A root of 5 plus a root of 7 by a root of 10 plus a root of 15 yields a root of 50, a root of 75, a root of 70, and a root of 105. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(\sqrt{5}+\sqrt{7}\right)\times\left(\sqrt{10}+\sqrt{15}\right)=\sqrt{50}+\sqrt{75}+\sqrt{70}+\sqrt{105}}}</math> | ||
| + | |style="text-align:right;"|שרש ה' ושרש ז' בשרש י' ושרש ט"ו עולה שרש נ' ושרש ע"ה ושרש ע' ושרש ק"ה | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== <span style=color:Green>Multiplication of Two Roots by Two other Roots - when the first roots of both parts are identical and the second roots of both parts are identical</span> ==== | ||
| + | |||
| + | | | ||
| + | |- | ||
| + | |If you wish to multiply a root plus a root by a root plus a root, when the later and the former numbers are identical. | ||
| + | |style="width:45%;text-align:right;"|<big>אם</big> בקשת לכפול שרש ושרש בשרש ושרש בהיות שוים המספרי' השניים והראשוני‫' | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:(√5+√7)×(√5+√7)|737|QSUQ}}Suppose you wish to multiply a root of 5 plus a root of 7 by a root of 5 plus a root of 7. | ||
| + | :<math>\scriptstyle\left(\sqrt{5}+\sqrt{7}\right)\times\left(\sqrt{5}+\sqrt{7}\right)</math> | ||
| + | |style="text-align:right;"|ונניח שרצית לכפול שרש ה' ושרש ז' בשרש ה' ושרש ז‫'{{#annotend:QSUQ}} | ||
| + | |- | ||
| + | | | ||
| + | :If you apply the way of the casillas, you should first multiply a root of 7 by a root of 7; the result is 7. Keep it. | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{7}\sdot\sqrt{7}=7}}</math> | ||
| + | |style="text-align:right;"|הנך צריך ראשנה אם באת לפעול בדרך הקאסילי לכפול שרש ז' בשרש ז' שעולה ז' ושומהו | ||
| + | |- | ||
| + | | | ||
| + | :Multiply a root of 7 by a root of 5 crosswise; the result is a root of 35. | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{7}\sdot\sqrt{5}=\sqrt{35}}}</math> | ||
| + | |style="text-align:right;"|אח"כ תכפול בשתי וערב שרש ז' בשרש ה' עולה שרש ל"ה | ||
| + | |- | ||
| + | | | ||
| + | :Then, multiply the other root of 7 by the other root of 5 crosswise; you get another root of 35. | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{7}\sdot\sqrt{5}=\sqrt{35}}}</math> | ||
| + | |style="text-align:right;"|ואח"כ תכפול שרש ז' האחר בשתי וערב בשרש ה' האחר ויהיה עוד לך שרש ל"ה | ||
| + | |- | ||
| + | | | ||
| + | :Sum these two roots with the 7 you kept; you get twice a root of 35, which is a root of 140, plus the 7 you have in your hand that you sum with them. | ||
| + | :<math>\scriptstyle{\color{blue}{7+\sqrt{35}+\sqrt{35}=7+2\sqrt{35}=\sqrt{140}+7}}</math> | ||
| + | |style="text-align:right;"|ואלו שני השרשי' תקבץ עם הז' ששמרת ויהיה לך שני פעמי' שרש ל"ה שהוא שרש ק"מ וז' יותר היו בידך אשר אשר תקבצם עמהם | ||
| + | |- | ||
| + | | | ||
| + | :Then, multiply a root of 5 by a root of 5; the result is 5. | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{5}\sdot\sqrt{5}=5}}</math> | ||
| + | |style="text-align:right;"|ואח"כ תכפול שרש ה' בשרש ה' ועולה ה‫' | ||
| + | |- | ||
| + | | | ||
| + | :Sum the 5 with the said sum; you get 12 plus a root of 140. | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{140}+7+5=12+\sqrt{140}}}</math> | ||
| + | |style="text-align:right;"|וזה הה' תקבץ עם הסך האמור ויהיה לך י"ב ושרש ק"מ | ||
| + | |- | ||
| + | | | ||
| + | :Even though we multiplied by the way of the casillas, we do not receive it by that way, because the first product is summed with the second in order to announce the numbers first and then the roots. | ||
| + | |style="text-align:right;"|ואפע"פ ש{{#annot:term|185,1230|PDGQ}}כפלנו בדרך הקסילי{{#annotend:PDGQ}} לא עלה לנו באופן ההוא מפני כי הכפל הראשון {{#annot:term|178,1841|9zb6}}קובץ עם{{#annotend:9zb6}} השני להשיב ראשונה המספרי' ואח"כ השרשי‫' | ||
| + | |- | ||
| + | | | ||
| + | :A root of 5 plus a root of 7 by a root of 5 plus a root of 7 yields a root of 140 plus the number 12. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(\sqrt{5}+\sqrt{7}\right)\times\left(\sqrt{5}+\sqrt{7}\right)=\sqrt{140}+12}}</math> | ||
| + | |style="text-align:right;"|שרש ה' ושרש ז' בשרש ה' ושרש ז' עולה שרש ק"מ וי"ב מספרי‫' | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== <span style=color:Green>Multiplication of a Root and a Root by a Root minus a Root</span> ==== | ||
| + | | | ||
| + | |- | ||
| + | |If you wish to multiply a root plus a root by a root minus a root: | ||
| + | |style="width:45%;text-align:right;"|<big>ואם</big> רצית לכפול שרש ושרש בשרש פחות שרש | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:(√5+√7)×(√10-√6)|737|fJYv}}Suppose you wish to multiply a root of 5 plus a root of 7 by a root of ten minus a root of 6. | ||
| + | :<math>\scriptstyle\left(\sqrt{5}+\sqrt{7}\right)\times\left(\sqrt{10}-\sqrt{6}\right)</math> | ||
| + | |style="text-align:right;"|ונניח שרצית לכפול שרש ה' ושרש ז' בשרש עשרה פחות שרש ו‫'{{#annotend:fJYv}} | ||
| + | |- | ||
| + | | | ||
| + | :Multiply first a root of 5 by a root of ten; the result is a root of 50. | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{5}\sdot\sqrt{10}=\sqrt{50}}}</math> | ||
| + | |style="text-align:right;"|תכפול ראשונה שרש ה' בשרש עשרה ועולה שרש נ‫' | ||
| + | |- | ||
| + | | | ||
| + | :Then, multiply crosswise a root of 7 that is additive by a root of ten; the result is a root of 70. | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{7}\sdot\sqrt{10}=\sqrt{70}}}</math> | ||
| + | |style="text-align:right;"|אח"כ תכה שרש שרש ז' שהוא יותר בשתי וערב בשרש עשרה ועולה שרש ע‫' | ||
| + | |- | ||
| + | | | ||
| + | :Sum [them] up; you get a root of 50 plus a root of 70. | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{50}+\sqrt{70}}}</math> | ||
| + | |style="text-align:right;"|ו{{#annot:term|178,1210|YVkR}}קבץ יחד{{#annotend:YVkR}} ויהיה לך שרש נ' ושרש ע‫' | ||
| + | |- | ||
| + | | | ||
| + | :Then, multiply crosswise [a root of 5 by] a subtractive root of 6; the result is a subtractive root of 30. | ||
| + | :<math>\scriptstyle{\color{blue}{{\color{red}{\sqrt{5}}}\sdot\left(-\sqrt{6}\right)=-\sqrt{30}}}</math> | ||
| + | |style="text-align:right;"|אח"כ {{#annot:term|2521,2522|EqX1}}תכה בשתי וערב{{#annotend:EqX1}} שרש ו' הפוחת ועושה שרש ל' הפוחת | ||
| + | |- | ||
| + | | | ||
| + | :Multiply a subtractive root of 6 by an additive root of 7; the result is a subtractive root of 42. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(-\sqrt{6}\right)\sdot\sqrt{7}=-\sqrt{42}}}</math> | ||
| + | |style="text-align:right;"|ואח"כ תכה שרש ו' הפוחת בשרש ז' המוסיף ועולה שרש מ"ב פוחת | ||
| + | |- | ||
| + | | | ||
| + | :Subtract these two subtractive products from the two former products; the remainder is a root of 50 plus a root of 70 minus a root of 30 minus a root of 42; this is the result of the multiplication of a root of 5 summed with a root of 7 by a root of ten minus a root of 6. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(\sqrt{5}+\sqrt{7}\right)\times\left(\sqrt{10}-\sqrt{6}\right)=\sqrt{50}+\sqrt{70}-\sqrt{30}-\sqrt{42}}}</math> | ||
| + | |style="text-align:right;"|ואלו שתי ההכאות הפוחתי' הוצא מהשתי הכאות ראשונות שעשית וישאר שרש נ' ושרש ע' פחות שרש ל' ופחות שרש מ"ב<br> | ||
| + | וככה עולה להכות שרש ה' {{#annot:term|178,1841|wUQf}}מקובץ עם{{#annotend:wUQf}} שרש ז' בשרש עשרה פחות שרש ו‫' | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== <span style=color:Green>Multiplication of a Root and a Root by a Root minus a Root - when the first roots of both parts are identical and the second roots of both parts are identical</span> ==== | ||
| + | |||
| + | | | ||
| + | |- | ||
| + | |If you wish to multiply a root plus a root by a root minus a root, when the first of the former is the same as the first of the [later] and the second of the former is the same as the second of the later. | ||
| + | |style="width:45%;text-align:right;"|<big>עוד</big> אם רצית לכפול שרש ושרש בשרש פחות שרש בהיות שוים הראשון מהחלק הראשון לראשון מהחלק הראשון והשני מהחלק ראשון לשני מהחלק השני | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:(√10+√7)×(√10-√7)|737|cbco}}Suppose you wish to multiply a root of ten plus a root of 7 by a root of ten minus a root of 7. | ||
| + | :<math>\scriptstyle\left(\sqrt{10}+\sqrt{7}\right)\times\left(\sqrt{10}-\sqrt{7}\right)</math> | ||
| + | |style="text-align:right;"|ונניח שרצית לכפול שרש עשרה ושרש ז' בשרש עשרה פחות שרש ז‫'{{#annotend:cbco}} | ||
| + | |- | ||
| + | |First, you should multiply the roots by each other: | ||
| + | |style="text-align:right;"|הנך צריך ראשונה לכפול השרשי' זה בזה | ||
| + | |- | ||
| + | | | ||
| + | :I.e. a root of ten by a root of ten; the result is ten. Keep it. | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{10}\sdot\sqrt{10}=10}}</math> | ||
| + | |style="text-align:right;"|דהיינו שרש עשרה בשרש עשרה ועולה עשרה ושמרם | ||
| + | |- | ||
| + | |Then, you only need to multiply the other roots by each other: | ||
| + | |style="text-align:right;"|אח"כ אין לך לכפול רק השרשי' האחרוני' זה בזה | ||
| + | |- | ||
| + | | | ||
| + | :I.e. a root of 7 by a subtractive root of 7; the result is a subtractive 7. | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{7}\sdot\left(-\sqrt{7}\right)=-7}}</math> | ||
| + | |style="text-align:right;"|דהיינו שרש ז' בפחות שרש ז' ועולה ז' הפוחת | ||
| + | |- | ||
| + | | | ||
| + | :Subtract the subtractive 7 from the ten you kept; three remains and this is the result of multiplication of a root of ten plus a root of seven by a root of ten minus a root of 7. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(\sqrt{10}+\sqrt{7}\right)\times\left(\sqrt{10}-\sqrt{7}\right)=10-7=3}}</math> | ||
| + | |style="text-align:right;"|והוצא זה הז' הפוחת מעשרה אשר שמרת ‫<ref>6v</ref>וישאר שלשה וככה עולה לכפול שרש עשרה ושרש ז' בשרש עשרה פחות שרש ז‫' | ||
| + | |- | ||
| + | |Remember that in this calculation and in similar [calculations] there is [no] need to multiply the roots crosswise, since they are the same - one is subtractive and the other is additive. | ||
| + | |style="text-align:right;"|וזכור כי בזה ה{{#annot:term|228,1200|E0MU}}חשבון{{#annotend:E0MU}} ובדומי' אליו צריך לכפול השרשי' בשתי וערב בהיותם שוים בהיות האחד פוחת והאחר מוסיף | ||
| + | |- | ||
| + | |Because one multiplication cancels the other, as one yields a subtractive and the other an additive. | ||
| + | |style="text-align:right;"|מפני כי הכפל האחד מכשיל האחר כי האחד עושה פחות והאחר יותר | ||
| + | |- | ||
| + | | | ||
| + | :So, when multiplying a root of ten plus a root of 7 by a root of ten minus a root of 7, the result is the number 3. | ||
| + | |style="text-align:right;"|והנה בכפול שרש עשרה ושרש ז' בשרש עשרה פחות שרש ז' עולה ג' מספרים | ||
| + | |- | ||
| + | |The same is done with all those that are similar to them. | ||
| + | |style="text-align:right;"|וכזה יעשה לכל הדומי' אליו | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== <span style=color:Green>Multiplication of a Root minus a Root by a Root minus a Root</span> ==== | ||
| + | | | ||
| + | |- | ||
| + | |If you wish to multiply a root minus a root by a root minus a root. | ||
| + | |style="width:45%;text-align:right;"|<big>עוד</big> אם רצית לכפול שרש פחות שרש בשרש פחות שרש | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:(√12-√7)×(√15-√10)|737|IWqT}}Suppose you wish to multiply a root of 12 minus a root of 7 by a root of 15 minus a root of ten. | ||
| + | :<math>\scriptstyle\left(\sqrt{12}-\sqrt{7}\right)\times\left(\sqrt{15}-\sqrt{10}\right)</math> | ||
| + | |style="text-align:right;"|נניח שרצית לכפול שרש י"ב פחות שרש ז' בשרש ט"ו פחות שרש עשרה{{#annotend:IWqT}} | ||
| + | |- | ||
| + | |First, you should multiply the additive roots by each other: | ||
| + | |style="text-align:right;"|תצטרך ראשונ' לכפול <s>השרשי'</s> השרשי' המוסיפי' זה בזה | ||
| + | |- | ||
| + | | | ||
| + | :I.e. a root of 12 by a root of 15; the result is a root of 180. Keep it. | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{12}\sdot\sqrt{15}=\sqrt{180}}}</math> | ||
| + | |style="text-align:right;"|דהיינו שרש י"ב בשרש ט"ו ועולה שרש ק"פ ושמרהו | ||
| + | |- | ||
| + | |Then, multiply the additive roots by the subtractive roots crosswise: | ||
| + | |style="text-align:right;"|אח"כ תכפול השרשי' המוסיפי' בשרשי' הגורעי' בשתי וערב | ||
| + | |- | ||
| + | | | ||
| + | :I.e. a root of 12 by a subtractive root of ten; the result is a root of 120 that is subtractive. | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{12}\sdot\left(-\sqrt{10}\right)=-\sqrt{120}}}</math> | ||
| + | |style="text-align:right;"|דהיינו שרש י"ב בפחות שרש עשרה ועולה שרש ק"כַ שהוא פחות | ||
| + | |- | ||
| + | | | ||
| + | :Multiply a root of 15 by a subtractive root of 7; the result is a root of 105 that is subtractive. | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{15}\sdot\left(-\sqrt{7}\right)=-\sqrt{105}}}</math> | ||
| + | |style="text-align:right;"|אח"כ תכפול שרש ט"ו בפחות שרש ז' ועולה שרש ק"ה שהוא פוחת | ||
| + | |- | ||
| + | |Set these two subtractive roots aside. | ||
| + | |style="text-align:right;"|ושים שני אלו השרשי' הפוחתי' לבד | ||
| + | |- | ||
| + | |Then, multiply the subtractive roots by each other: | ||
| + | |style="text-align:right;"|ואח"כ תכפול השרשי' הפוחתי' זה בזה | ||
| + | |- | ||
| + | | | ||
| + | :I.e. a root of 7 by a root of ten; the result is a root of 70 that is additive. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(-\sqrt{7}\right)\sdot\left(-\sqrt{10}\right)=\sqrt{70}}}</math> | ||
| + | |style="text-align:right;"|דהיינו שרש ז' בשרש עשרה ועולה שרש ע' שהוא יותר | ||
| + | |- | ||
| + | | | ||
| + | :Add it to the additive product you have; you get a root of 180 plus a root of 70. | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{180}+\sqrt{70}}}</math> | ||
| + | |style="text-align:right;"|<s>וזה</s> <sup>ואלו</sup> תוסיפם עם הכפל שעשית יותר ויהיה לך שרש ק"פ ושרש ע' <s>פחות שרש ק"כ</s> | ||
| + | |- | ||
| + | | | ||
| + | :Now, subtract the two subtractive roots from this sum; a root of 180 plus a root of 70 remains minus a root of 120 minus a root of 105 and this is the result of the mentioned multiplication. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(\sqrt{12}-\sqrt{7}\right)\times\left(\sqrt{15}-\sqrt{10}\right)=\sqrt{180}+\sqrt{70}-\sqrt{120}-\sqrt{105}}}</math> | ||
| + | |style="text-align:right;"|עתה הוצא שני השרשי' הפוחתי' מזה הסך וישאר שרש ק"פ ושרש ע' פחות שרש ק"כ ופחות שרש ק"ה וככה עולה הכפל הנז‫' | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== <span style=color:Green>Multiplication of a Root minus a Root by a Root minus a Root - when the first roots of both parts are identical and the second roots of both parts are identical</span> ==== | ||
| + | |||
| + | | | ||
| + | |- | ||
| + | |If you wish to multiply a root minus a root by a root minus a root, when the first [of the former] is the same as the first [of the later] and the second [of the former] is the same as the second of the later. | ||
| + | |style="width:45%;text-align:right;"|<big>עוד</big> אם רצית לכפול שרש פחות שרש בשרש פחות שרש בהיות שוים השרשי' הראשוני' לראשוני' והשניים לשניים מהחלק האחר | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:(√12-√7)×(√12-√7)|737|ISCj}}Suppose you wish to multiply a root of 12 minus a root of 7 by a root of 12 minus a root of 7. | ||
| + | :<math>\scriptstyle\left(\sqrt{12}-\sqrt{7}\right)\times\left(\sqrt{12}-\sqrt{7}\right)</math> | ||
| + | |style="text-align:right;"|ונניח שרצית לכפול שרש י"ב פחות שרש ז' בשרש י"ב פחות שרש ז‫'{{#annotend:ISCj}} | ||
| + | |- | ||
| + | |First, you should multiply the [additive] roots by each other: | ||
| + | |style="text-align:right;"|אתה צריך ראשונה לכפול השרשי' הרבי' זה בזה | ||
| + | |- | ||
| + | | | ||
| + | :I.e. a root of 12 by a root of 12; the result is 12. Keep it. | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{12}\sdot\sqrt{12}=12}}</math> | ||
| + | |style="text-align:right;"|דהיינו שרש י"ב בשרש י"ב ועולה י"ב ושמרם | ||
| + | |- | ||
| + | |Then, multiply the [subtractive] roots by each other: | ||
| + | |style="text-align:right;"|אח"כ תכפול השרשי' שהם מעטי' זה בזה | ||
| + | |- | ||
| + | | | ||
| + | :I.e. a subtractive root of 7 by a subtractive root of 7; the result is an additive 7. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(-\sqrt{7}\right)\sdot\left(-\sqrt{7}\right)=7}}</math> | ||
| + | |style="text-align:right;"|דהיינו הפוחת שרש ז' בפוחת שרש ז' ועולה ז' יותר | ||
| + | |- | ||
| + | | | ||
| + | :Sum it with what you kept; you have 19. Keep it. | ||
| + | :<math>\scriptstyle{\color{blue}{12+7=19}}</math> | ||
| + | |style="text-align:right;"|וקבצם יחד עם אשר שמרת ויהיה לך י"ט ושמרם | ||
| + | |- | ||
| + | |Then, multiply the additive roots by the [subtractive] roots crosswise: | ||
| + | |style="text-align:right;"|אח"כ תכה השרשי' שהם יותר בשרשי' שהם מעט בשתי וערב | ||
| + | |- | ||
| + | | | ||
| + | :I.e. a root of 12 by a subtractive root of 7 and a subtractive root of 7 by a root of 12; you have twice a root of 84. These two roots are 2 multiplied by a root of 84; the result is a subtractive root of 336. | ||
| + | :<math>\scriptstyle{\color{blue}{\left[\sqrt{12}\sdot\left(-\sqrt{7}\right)\right]+\left[\left(-\sqrt{7}\right)\sdot\sqrt{12}\right]=2\sdot\left({\color{red}{-}}\sqrt{84}\right)=-\sqrt{336}}}</math> | ||
| + | |style="text-align:right;"|דהיינו שרש י"ב בשרש ז' גורעי' ושרש ז' הגורע עוד בשרש י"ב ויהיה לך ב' פעמי' שרש פ"ד אשר שני אלו השרשי' הם ב' <s>בש</s> מוכה בשרש פ"ד שעולה שרש של"ו גורעי‫' | ||
| + | |- | ||
| + | | | ||
| + | :Subtract it from the sum you kept, which is 19; 19 minus a root of 336 remains and this is the result of multiplication of a root of 12 minus a root of 7 by a root of 12 minus a root of 7. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(\sqrt{12}-\sqrt{7}\right)\times\left(\sqrt{12}-\sqrt{7}\right)=19-\sqrt{336}}}</math> | ||
| + | |style="text-align:right;"|הוציאם מהסך ששמרת שהוא י"ט ו{{#annot:term|936,1236|a2Jj}}ישאר לך{{#annotend:a2Jj}} י"ט פחות שרש של"ו וככה עולה לכפול שרש י"ב פחות שרש ז' בשרש י"ב פחות שרש ז‫' | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== <span style=color:Green>Multiplication of a Number by a Root</span> ==== | ||
| + | | | ||
| + | |- | ||
| + | |Remember that whenever you happen to multiply a number by a root, you should convert the number into a root of the same degree - whether a square root, or a cube root, or any other degree. | ||
| + | |style="width:45%;text-align:right;"|<big>וזכור</big> כי בכל פעם שיגיע בידך לכפול מספר באיזה שרש <sup>צריך</sup> ש{{#annot:term|1071,1431|Pvy2}}תשיב המספר למין השרש{{#annotend:Pvy2}} אשר אתה רוצה לכפול מרובע או מעוקב או בכל אופן שיוכל להגיע | ||
| + | |- | ||
| + | |If you happen to multiply a root by another root of a different degree [lit. not similar to it by nature], you should convert each of the roots to the root of the parallel degree. | ||
| + | |style="text-align:right;"|ואם יגיע בידך לכפול איזה שרש בשרש אחר אינו דומה אליו בטבע אתה צריך להשיב כל אחד ממספרי השרשי' ההם אל שרש המספר המתנגד לטבע | ||
| + | |- | ||
| + | | | ||
| + | :For instance - a number by roots: when a number is multiplied by a square root, the number should be multiplied by itself, then multiply the product by the other root; the root of the result is their product, as the method explained above. | ||
| + | :<math>\scriptstyle\scriptstyle{\color{OliveGreen}{a\times\sqrt{b}=\sqrt{a^2\sdot b}}}</math> | ||
| + | |style="text-align:right;"|והנה המשל מהמספר בשרשי' בכפול מספר בשרש מרובע צריך לכפול המספר בעצמו ואח"כ תכפול העולה ממנו במספר השרש האחר והעולה מזה הנה שרשו הוא יהיה הכאתו כאשר יתבאר לפנים בזה האופן | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:3×√4|737|eTRG}}Suppose you want to multiply 3 by a square root of 4. | ||
| + | :<math>\scriptstyle3\times\sqrt{4}</math> | ||
| + | |style="text-align:right;"|ונניח שבאת ‫<ref>7r</ref>לכפול ג' בשרש מרובע מד‫'{{#annotend:eTRG}} | ||
| + | |- | ||
| + | | | ||
| + | :You should convert 3 into a square root, which is a root of 9. | ||
| + | :<math>\scriptstyle{\color{blue}{3=\sqrt{9}}}</math> | ||
| + | |style="text-align:right;"|הנך צריך להשיב ג' לשרש מרובע שהוא שרש מט‫' | ||
| + | |- | ||
| + | | | ||
| + | :Multiply 4 by 9; it yields 36. | ||
| + | :<math>\scriptstyle{\color{blue}{4\sdot9=36}}</math> | ||
| + | |style="text-align:right;"|ותכפול ד' בט' שעושה ל"ו | ||
| + | |- | ||
| + | | | ||
| + | :A root of 36, which is 6, is the said product. | ||
| + | :<math>\scriptstyle{\color{blue}{3\times\sqrt{4}=\sqrt{36}=6}}</math> | ||
| + | |style="text-align:right;"|ושרש ל"ו שהוא ו' הוא הכפל האמור | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:3׳√8|737|O0bJ}}If you multiply 3 by a cube root of 8. | ||
| + | :<math>\scriptstyle3\times\sqrt[3]{8}</math> | ||
| + | |style="text-align:right;"|<big>ואם</big> תכפול ג' בשרש מעוקב מח‫'{{#annotend:O0bJ}} | ||
| + | |- | ||
| + | | | ||
| + | :You should convert 3 into a cube root, which is a cube root of 27. | ||
| + | :<math>\scriptstyle{\color{blue}{3=\sqrt[3]{27}}}</math> | ||
| + | |style="text-align:right;"|אתה צריך להשיב ג' לשרש מעוקב שהוא יהיה שרש מעוקב מכ"ז | ||
| + | |- | ||
| + | | | ||
| + | :Multiply 8 by 27; the result is 216. | ||
| + | :<math>\scriptstyle{\color{blue}{8\sdot27=216}}</math> | ||
| + | |style="text-align:right;"|ותכפול ח' בכ"ז ועולה רי"ו | ||
| + | |- | ||
| + | | | ||
| + | :A cube root of 216, which is 6, is the said product. | ||
| + | :<math>\scriptstyle{\color{blue}{3\times\sqrt[3]{8}=\sqrt[3]{216}=6}}</math> | ||
| + | |style="text-align:right;"|ושרש מעוקב מרי"ו שהוא ו' הוא הכפל האמור | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== <span style=color:Green>Multiplication of a Square Root by a Cubic Root</span> ==== | ||
| + | | | ||
| + | |- | ||
| + | |If you happen to multiply a square root by a cube root. | ||
| + | |style="width:45%;text-align:right;"|<big>ואם</big> יקרה לך לכפול שרש מרובע בשרש מעוקב | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:√4׳√8|737|J15w}}Suppose you wish to multiply a square root of 4 by a cube root of 8. | ||
| + | :<math>\scriptstyle\sqrt{4}\times\sqrt[3]{8}</math> | ||
| + | |style="text-align:right;"|נניח שרצית לכפול שרש מרובע מד' בשרש מעוקב מח‫'{{#annotend:J15w}} | ||
| + | |- | ||
| + | | | ||
| + | :You should convert 4 into a cube root; you get a cube root of 64. | ||
| + | :<math>\scriptstyle{\color{blue}{4=\sqrt[3]{64}}}</math> | ||
| + | |style="text-align:right;"|הנך צריך להשיב ד' לשרש מעוקב ויהיה לך שרש מעוקב מס"ד | ||
| + | |- | ||
| + | | | ||
| + | :Then, convert 8 into a root; you get a square root of 64. | ||
| + | :<math>\scriptstyle{\color{blue}{8=\sqrt{64}}}</math> | ||
| + | |style="text-align:right;"|אח"כ תשיב ח' אל שרשי' ויהיה לך שרש ס"ד מרובע | ||
| + | |- | ||
| + | | | ||
| + | :Now, multiply a square root of a cube root of 64 by a square root of a cube root of 64, or by a cube root of a square root of 64. | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{\sqrt[3]{64}}\sdot\sqrt{\sqrt[3]{64}}=\sqrt{\sqrt[3]{64}}\times\sqrt[3]{\sqrt{64}}}}</math> | ||
| + | |style="text-align:right;"|עתה תכפול {{#annot:term|2634|PC05}}שרש מרובע משרש מעוק' מ{{#annotend:PC05}}ס"ד <s>ויהיה לך</s> בשרש מרובע משרש מעוקב מס"ד או ב{{#annot:term|2634|r7zL}}שרש מעוקב משרש מרובע מ{{#annotend:r7zL}}ס"ד | ||
| + | |- | ||
| + | |It can be expressed by the first way or the other. | ||
| + | |style="text-align:right;"|כי יתכן לומר באופן האחד כמו באחר | ||
| + | |- | ||
| + | | | ||
| + | :I.e. 64 by 64; the result is 4096. | ||
| + | :<math>\scriptstyle{\color{blue}{64\sdot64=4096}}</math> | ||
| + | |style="text-align:right;"|דהיינו ס"ד בס"ד ועולה ד' אלפי' וצ"ו | ||
| + | |- | ||
| + | | | ||
| + | :The square root of the cube root, or say, the cube root of the square root of 4096 is the said product and it is 4. | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{4}\times\sqrt[3]{8}=\sqrt{\sqrt[3]{4096}}=\sqrt[3]{\sqrt{4096}}=4}}</math> | ||
| + | |style="text-align:right;"|ו{{#annot:term|2634|ZtBh}}שרש מרובע מהשרש מעוקב{{#annotend:ZtBh}} או תאמ' {{#annot:term|2634|mNas}}השרש המעוקב משרש המרובע מ{{#annotend:mNas}}ד' אלפי' וצ"ו הוא הכפל האמור והוא ד‫' | ||
| + | |- | ||
| + | | | ||
| + | :3 by a square root of 4, i.e. a root of 9 by a root of 4, yields a root of 36, which is 6. | ||
| + | :<math>\scriptstyle{\color{blue}{3\times\sqrt{4}=\sqrt{9}\times\sqrt{4}=\sqrt{36}=6}}</math> | ||
| + | |style="text-align:right;"|ג' בשרש מרובע מד' דהיינו שרש ט' בשרש ד' עולה שרש מל"ו שהוא ו‫' | ||
| + | |- | ||
| + | | | ||
| + | :3 by a cube root of 8, which is a cube root of 27 by a cube root of 8, yields a cube root of 216, which is 6. | ||
| + | :<math>\scriptstyle{\color{blue}{3\times\sqrt[3]{8}=\sqrt[3]{27}\times\sqrt[3]{8}=\sqrt[3]{216}=6}}</math> | ||
| + | |style="text-align:right;"|ג' בשרש מעוקב מח' שהוא שרש מעו' מכ"ז בשרש מעו' מח' עולה שרש מעו' מרי"ו והוא ו‫' | ||
| + | |- | ||
| + | | | ||
| + | :A square root of 4 by a cube root of 8 yields a square root of a cube root of 4096. | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{4}\times\sqrt[3]{8}=\sqrt{\sqrt[3]{4096}}}}</math> | ||
| + | |style="text-align:right;"|שרש מרובע מד' בשרש מעוקב מח' עולה שרש מרובע משרש מעו' <s>מאלף</s> מד' אלפי' וצ"ו | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== <span style=color:Green>Multiplication of a Cube Root by a Root of a Root</span> ==== | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:³√8×⁴√16|737|ek3B}}If you are told: multiply a cube root of 8 by a root of a root of 16. | ||
| + | :<math>\scriptstyle\sqrt[3]{8}\times\sqrt[4]{16}</math> | ||
| + | |style="text-align:right;"|<big>עוד</big> אם יאמרו לך תכפול שרש מעוקב מח' בשרש שרש י"ו{{#annotend:ek3B}} | ||
| + | |- | ||
| + | |Convert the number in the cube root to a root of a root, and the number in the root of the root to a cube root. | ||
| + | |style="width:45%;text-align:right;"|השב מספר השרש מעוקב לשרש השרש <s>מהשרש</s> ומספר שרש השרש למעוקב | ||
| + | |- | ||
| + | |Then, multiply them by each other, and the cube root of the root of the root, or the root of the root of the cube root of the result is the stated product. | ||
| + | |style="text-align:right;"|ואח"כ תכפול זה בזה ושרש <s>מקו</s> מעוקב שרש השרש או שרש שרש המעוקב ההוה הוא יהיה הכפל האמור | ||
| + | |- | ||
| + | | | ||
| + | :In the example, [convert] 8 into a root of a root: say 8 multiplied by 8 yields 64, and 64 multiplied by 64 yields 4096. | ||
| + | :<math>\scriptstyle{\color{blue}{8^4=\left(8\sdot8\right)^2=64^2=4096}}</math> | ||
| + | |style="text-align:right;"|והנה הדמיון תכפול ח' באופן שרש השרש ואמור ח' מוכה בח' עושה ס"ד וס"ד מוכה בס"ד עולה <s>אלף וצ"ו</s> ד' אלפים וצ"ו | ||
| + | |- | ||
| + | | | ||
| + | :Then, multiply 16 cubically: say 16 multiplied by 16 yields 256. Say 16 multiplied by 256 that is the cube yields 4096. | ||
| + | :<math>\scriptstyle{\color{blue}{16^3=\left(16\sdot16\right)\sdot16=256\sdot16=4096}}</math> | ||
| + | |style="text-align:right;"|אח"כ תכה י"ו באופן מעוקב ואמור י"ו מוכה בי"ו עולה רנ"ו ואח"כ אמור י"ו מוכה ברנ"ו שהוא המעוקב ועולה ד' אלפי' וצ"ו | ||
| + | |- | ||
| + | | | ||
| + | :Multiply these numbers by each other, which is 4096 by 4096; the result is 16777216. | ||
| + | :<math>\scriptstyle{\color{blue}{4096\sdot4096=16777216}}</math> | ||
| + | |style="text-align:right;"|ואלו המספרי' תכפול זה בזה והם ד' אלפי' וצ"ו בד' אלפי' וצ"ו ועלו 16777216 | ||
| + | |- | ||
| + | | | ||
| + | :The cube root of the root of the root of the said number, or say the root of the root of the cube root of the stated number, is the result of multiplication of a cube root of 8 by a root of a root of 16. The result is 4. | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt[3]{8}\times\sqrt[4]{16}=\sqrt[3]{\sqrt[4]{16777216}}=\sqrt[4]{\sqrt[3]{16777216}}=4}}</math> | ||
| + | |style="text-align:right;"|ו{{#annot:term|2634|K6Hj}}השרש מעוקב משרש השרש מ{{#annotend:K6Hj}}הסך האמור או נאמ' {{#annot:term|2634|oDfj}}שרש שרש מהשרש המעוקב מ{{#annotend:oDfj}}הסך האמור עולה בכפול שרש מעוקב מח' בשרש שרש י"ו העולה ד' במספר | ||
| + | |- | ||
| + | | | ||
| + | :A cube root of 8 multiplied by a root of a root of 16 is a cube root of a root of a root or a root of a root of a cube root of 16777216, which is 4. | ||
| + | |style="text-align:right;"|שרש מעוקב מח' מוכה בשרש משרש י"ו שרש מעו' משרש שרש או שרש משרש משרש שרש מעוקב מ 16777216 שהוא ד‫' | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== <span style=color:Green>Multiplication of a Number and a Root of a Number and a Root by a Number and a Root of a Number and a Root</span> ==== | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | *If you wish to multiply a half plus a root of a sum of a quarter and a root of 12 by a half plus a root of a sum of a quarter and a root of 12. | ||
| + | :<math>\scriptstyle\left(\frac{1}{2}+\sqrt{\frac{1}{4}+\sqrt{12}}\right)\times\left(\frac{1}{2}+\sqrt{\frac{1}{4}+\sqrt{12}}\right)</math> | ||
| + | |style="text-align:right;"|<big>עוד</big> אם רצית לכפול חצי ושרש מ{{#annot:term|388,1213|tBcJ}}נוסף{{#annotend:tBcJ}} {{#annot:term|388|az6A}}זינטו{{#annotend:az6A}} בלעז רביע אחד עם שרש י"ב בחצי אחד ושרש מ{{#annot:term|788,1213|Clw0}}נוסף{{#annotend:Clw0}} רביע אחד עם שרש י"ב | ||
| + | |- | ||
| + | | | ||
| + | :First, you should multiply a half by a half; it yields a quarter. Keep it. | ||
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{2}\sdot\frac{1}{2}=\frac{1}{4}}}</math> | ||
| + | |style="width:45%;text-align:right;"|‫<ref>7v</ref>אתה צריך ראשונה לכפול חצי בחצי ועושה רביע ושמרהו | ||
| + | |- | ||
| + | | | ||
| + | :Then, multiply a half by a root of a sum of a quarter and a root of 12; the result is a root of a sum of 1 [part] of 16 and a root of 3-quarters. | ||
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{2}\sdot\sqrt{\frac{1}{4}+\sqrt{12}}=\sqrt{\frac{1}{16}+\sqrt{\frac{3}{4}}}}}</math> | ||
| + | |style="text-align:right;"|אח"כ תכפול חצי בשרש מנוסף רביע עם שרש י"ב ועולה שרש מנוסף א' מי"ו עם שרש ג' רביעי‫' | ||
| + | |- | ||
| + | | | ||
| + | :Multiply also a half by a root of a sum of a quarter and a root of 12 crosswise; the result is a root of a sum of one part of 16 and a root of 3-quarters. | ||
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{2}\sdot\sqrt{\frac{1}{4}+\sqrt{12}}=\sqrt{\frac{1}{16}+\sqrt{\frac{3}{4}}}}}</math> | ||
| + | |style="text-align:right;"|אח"כ תכפול עוד בשתי וערב חצי בשרש מנוסף רביע עם שרש י"ב ועולה שרש מנוסף חלק מי"ו עם שרש ג' רביעי‫' | ||
| + | |- | ||
| + | | | ||
| + | :These two identical products are as if you say 2 by a root of a sum of one part of 16 and a root of 3-quarters; the result is a root of a sum of a quarter and a root of 12. Keep it. | ||
| + | :<math>\scriptstyle{\color{blue}{2\sdot\sqrt{\frac{1}{16}+\sqrt{\frac{3}{4}}}=\sqrt{\frac{1}{4}+\sqrt{12}}}}</math> | ||
| + | |style="text-align:right;"|ואלו שני הכפלי' השוים הם כאלו אמרת ב' בשרש מנוסף חלק מי"ו עם שרש ג' רביעי' שעולה שרש מנוסף רביע עם שרש י"ב ושמור | ||
| + | |- | ||
| + | | | ||
| + | :Then, multiply a root of a sum of a quarter and a root of 12 by a root of a sum of a quarter and a root of 12; the result is a quarter and a root of 12. | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{\frac{1}{4}+\sqrt{12}}\sdot\sqrt{\frac{1}{4}+\sqrt{12}}=\frac{1}{4}+\sqrt{12}}}</math> | ||
| + | |style="text-align:right;"|אח"כ תכפול שרש מנוסף רביע עם שרש י"ב בשרש מנוסף רביע עם שרש י"ב שעולה רביע אחד ושרש מי"ב | ||
| + | |- | ||
| + | | | ||
| + | :Now, sum up all these products together; the result is one half and a root of 12, plus a root of a sum of a quarter and a root of 12. | ||
| + | |style="text-align:right;"|עתה תקבץ כל אלו ה{{#annot:term|241,1253|gaGU}}כפילות{{#annotend:gaGU}} יחד ו{{#annot:term|875,1240|AULn}}עולות{{#annotend:AULn}} חצי אחד ושרש מי"ב ויותר שרש מנוסף רביע עם שרש מי"ב | ||
| + | |- | ||
| + | | | ||
| + | :This is the result of the said multiplication: a half plus a root of a sum of a quarter and a root of 12 by a half plus a root of a sum of a quarter and a root of 12; the result is one half and a root of 12, plus a root of a sum of a quarter and a root of 12. | ||
| + | |style="text-align:right;"|וככה עולה הכפל האמור חצי ושרש מנוסף רביע עם שרש י"ב בחצי ושרש מנוסף רביע עם שרש מי"ב עולה חצי אחד ושרש מי"ב ושרש מנוסף רביע אחד עם שרש מי"ב | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{\left(\frac{1}{2}+\sqrt{\frac{1}{4}+\sqrt{12}}\right)\times\left(\frac{1}{2}+\sqrt{\frac{1}{4}+\sqrt{12}}\right)=\frac{1}{2}+\sqrt{12}+\sqrt{\frac{1}{4}+\sqrt{12}}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :Know that when you multiply the number by a part of [the other] number - i.e. a half by the root of the two parts that are summed together, i.e. a quarter an a root of 12 - you should convert the half into a root. You have a root of a quarter. | ||
| + | |style="text-align:right;"|<big>ודע</big> כי כאשר תכפול המספר עם החלק מהמספר דהיינו חצי בשרש שני החלקי' {{#annot:term|178,1213|SkZ4}}הנוספים יחד{{#annotend:SkZ4}} דהיינו א' רביע {{#annot:term|178,1213|Pza2}}נוסף עם{{#annotend:Pza2}} שרש מי"ב אתה צריך להשיב החצי אל שרש ויהיה לך שרש [מא'] רביע | ||
| + | |- | ||
| + | | | ||
| + | :Multiply this quarter by the quarter that is summed with a root of 12; the result is one part of 16. | ||
| + | |style="text-align:right;"|ותכפול זה הרביע ברביע הנוסף עם שרש י"ב ועולה חלק אחד מי"ו | ||
| + | |- | ||
| + | | | ||
| + | :Then, convert the half mentioned into a root of a root; you get a root of a root of one part of 16. | ||
| + | |style="text-align:right;"|אח"כ {{#annot:term|1071,1431|qStG}}השב ה{{#annotend:qStG}}חצי האמור אל שרש משרש ויהיה לך שרש משרש מחלק מי"ו | ||
| + | |- | ||
| + | | | ||
| + | :Multiply this part of 16 by 12, which is the radicand; the result is 3-quarters. | ||
| + | |style="text-align:right;"|וזה החלק מי"ו תכפול בי"ב שהוא המספר {{#annot:term|1073,2300|jxnc}}הנקוב <sup>בשם</sup> שיש לו שרש{{#annotend:jxnc}} ועולה ג' רביעים | ||
| + | |- | ||
| + | | | ||
| + | :Sum up this product in one expression similar to the written above; you get a root of a sum [of one part of 16] and a root of 3-quarters. | ||
| + | |style="text-align:right;"|וקבץ זה הכפל יחד בקול דומה לאשר הוא כתוב לפנים ויהיה לך שרש נוסף עם שרש מג' רביעי‫' | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{2}\sdot\sqrt{\frac{1}{4}+\sqrt{12}}=\sqrt{\left(\frac{1}{4}\sdot\frac{1}{4}\right)+\left(\frac{1}{16}\sdot\sqrt{12}\right)}=\sqrt{\frac{1}{16}+\sqrt{\frac{1}{16}\sdot12}}=\sqrt{\frac{1}{16}+\sqrt{\frac{3}{4}}}}}</math> | ||
| + | |- | ||
| + | |Then, perform the other similar multiplication crosswise. | ||
| + | |style="text-align:right;"|ואח"כ תעשה בשתי וערב הכפל האחר הדומה לזה | ||
| + | |- | ||
| + | | | ||
| + | :You get this multiplication twice, which is two by a root of a sum of one part of 16 and a root of 3-quarters. | ||
| + | :<math>\scriptstyle{\color{blue}{2\sdot\sqrt{\frac{1}{16}+\sqrt{\frac{3}{4}}}}}</math> | ||
| + | |style="text-align:right;"|ויהיה לך הכפל הזה כפול אשר יהיה שנים בשרש הנוסף חלק מי"ו עם שרש ג' רביעי‫' | ||
| + | |- | ||
| + | | | ||
| + | :Therefore, you should convert the two into a root also; the result is 4. | ||
| + | |style="text-align:right;"|ובגלל זה אתה צריך להשיב כמו כן זה השנים אל שרש ועולה ד‫' | ||
| + | |- | ||
| + | | | ||
| + | :Multiply the 4 by one part of 16; the result is one part of 4. | ||
| + | |style="text-align:right;"|וזה הד' תכפול בחלק מי"ו שעולה חלק מד‫' | ||
| + | |- | ||
| + | | | ||
| + | :Then, convert the 2 mentioned into a root of a root; you get 16. | ||
| + | |style="text-align:right;"|אח"כ השב הב' האמור לשרש משרש ויהיה לך י"ו | ||
| + | |- | ||
| + | | | ||
| + | :Multiply the 16 by 3-quarters; the result is 12. | ||
| + | |style="text-align:right;"|וזה הי"ו תכפול בג' רביעים ועולה י"ב | ||
| + | |- | ||
| + | | | ||
| + | :This product is summed with the expression of the above part of the product; you get a root of a sum of a quarter and a root of 12. | ||
| + | |style="text-align:right;"|וזה הכפל מקובץ יחד כפי הקול מהחלק מהכפל האמור ויהיה לך שרש מנוסף א' רביע עם שרש מי"ב | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{2\sdot\sqrt{\frac{1}{16}+\sqrt{\frac{3}{4}}}=\sqrt{\left(2^2\sdot\frac{1}{16}\right)+\sqrt{2^4\sdot\frac{3}{4}}}=\sqrt{\left(4\sdot\frac{1}{16}\right)+\sqrt{16\sdot\frac{3}{4}}}=\sqrt{\frac{1}{4}+\sqrt{12}}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :This is the result of the crosswise multiplication. | ||
| + | |style="text-align:right;"|וככה עולה החלק מהכפל העשוי בשתי וערב | ||
| + | |- | ||
| + | |Now multiply the other multiplicands by themselves as said above, in order to complete the multiplication. | ||
| + | |style="text-align:right;"|עתה תכפול החלקי' האחרים בעצמם כפי האמור למעלה להשלים הכפל | ||
| + | |- | ||
| + | |The reason you convert the number into a root, then into a root of the root: | ||
| + | |style="text-align:right;"|והסבה שאתה תשיב המספר אל שרש ואח"כ אל שרש השרש | ||
| + | |- | ||
| + | |Because you multiply it by the root of the number that is added to a root of a number. | ||
| + | |style="text-align:right;"|<s>הוא</s> הוא בגלל שאתה כופלו בשרש {{#annot:term|787,1213|rt6p}}המספר הנוסף{{#annotend:rt6p}} עם שרש מספר | ||
| + | |- | ||
| + | | | ||
| + | :That is by a root of the sum of a quarter and a root of 12, which is as you multiply 2 by a root of the sum of a part of 16 and a root of 3-quarters. | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{\frac{1}{4}+\sqrt{12}}=2\sdot\sqrt{\frac{1}{16}+\sqrt{\frac{3}{4}}}}}</math> | ||
| + | |style="text-align:right;"|שהוא בשרש הנוסף רביע עם שרש מי"ב הוא כאלו כפלת ב' בשרש מנוסף חלק מי"ו עם שרש מג' רביעים | ||
| + | |- | ||
| + | | | ||
| + | :You convert the 2 into a root, in order to multiply it by the root of the additive number, then you convert it into a root of a root, in order to multiply it by the root of the root of the additive number. | ||
| + | |style="text-align:right;"|אתה {{#annot:term|1071,1431|f1HX}}השיבות ה{{#annotend:f1HX}}ב' אל שרש לכפלו עם שרש {{#annot:term|788,1213|PUZW}}המספר הנוסף {{#annotend:PUZW}}ואח"כ השיבות אותו לשרש משרש לכפלו <s>ש</s> בשרש השרש מהמספר הנוסף | ||
| + | |- | ||
| + | | | ||
| + | :Similarly to what you did with the half , because you do with the 2 as you saw in the example. | ||
| + | |style="text-align:right;"|<s>ג"כ</s> בדומה לאשר עשית מהחצי כי עשית מהב' כאשר ראית בדמיון | ||
| + | |- | ||
| + | | | ||
| + | :A half plus a root of a sum of a quarter and a root of 12 by a half plus a root of a sum of a quarter and a root of 12; the result is a half and a root of 12 plus a root of a sum of a quarter and a root of 12. | ||
| + | |style="text-align:right;"|חצי ושרש מנוסף רביע עם שרש י"ב בחצי ושרש מנוסף רביע עם שרש י"ב עולה <s>חצי ושרש מנוסף רביע על שרש י"ב</s> חצי ושרש מי"ב ושרש מנוסף רביע עם שרש מי"ב | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{\left(\frac{1}{2}+\sqrt{\frac{1}{4}+\sqrt{12}}\right)\times\left(\frac{1}{2}+\sqrt{\frac{1}{4}+\sqrt{12}}\right)=\frac{1}{2}+\sqrt{12}+\sqrt{\frac{1}{4}+\sqrt{12}}}}</math> | ||
| + | |- | ||
| + | |Know that many other multiplications can fall into your hands. | ||
| + | |style="text-align:right;"|‫<ref>8r</ref><big>ודע</big> כי {{#annot:term|156,1230|0vQW}}כפלים{{#annotend:0vQW}} אחרים רבים שונים מאלו יוכל להגיע לידך | ||
| + | |- | ||
| + | |They have no end, therefore it is impossible to write rules for all of them. | ||
| + | |style="text-align:right;"|ואין סוף להם ולכן לא יתכן לכתוב כללים לכלם | ||
| + | |- | ||
| + | |Yet, from the aforesaid rules it is possible to understand and to present the rule, according to the aforesaid teaching, for every multiplication that comes, or that may come. | ||
| + | |style="text-align:right;"|אבל מן הכללים האמורים יתכן להבין ולתת כלל כפי הלמוד האמור לכל כפל שיגיע ושיוכל לבא | ||
| + | |- | ||
| + | |Since, when multiplying the roots, one answers by saying the sum of a root of this and a root of that. | ||
| + | |style="text-align:right;"|<big>בהיות</big> כי בכפול השרשי' תעשה תשובה בהאמר בסך שרש מכך ושרש מכך | ||
| + | |- | ||
| + | |Many times two or three or more types are summed, when a part of another part results from the multiplication, or when they cannot be summed together in one expression. | ||
| + | |style="text-align:right;"|ופעמי' רבים ב' או ג' מינים ויותר {{#annot:term|178,1219|l0Bm}}נקבצים{{#annotend:l0Bm}} כאשר יעלו מהכפל הנעשה חלק אחר חלק וכאשר לא יתחברו יחד בקול אחד | ||
| + | |- | ||
| + | |Except for the roots that are equal and summed by doubling and duplicating: | ||
| + | |style="text-align:right;"|לבד השרשי' אשר היו שוים ונתוספו באופן הכפל וההכפלה | ||
| + | |- | ||
| + | |i.e. since when there are two equal roots, multiplying one of them by two yields the same as summing them together. <math>\scriptstyle2\sdot\sqrt{a}=\sqrt{a}+\sqrt{a}</math> | ||
| + | |style="text-align:right;"|דהיינו כי בהיות שני שרשי' שוים {{#annot:term|358,2136|rxHJ}}בהכפל{{#annotend:rxHJ}} אחד מהם בשנים עושה כך כמו בחברם יחד | ||
| + | |- | ||
| + | |and if there were three equal [roots] - multiplying one of them by three | ||
| + | |style="text-align:right;"|ואם היו ג' שוים בהכפל אחד מהם בג‫' | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|וג"כ בהיות מינים יותר {{#annot:term|185,1622|nXdE}}בהכפילם ב{{#annotend:nXdE}}כל כך מספר כמו שהם השרשי' השוים עושה כך כמו מחוברי' יחד | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | === <span style=color:Green>Addition and Subtraction of Roots</span> === | ||
| + | |||
| + | | | ||
| + | |- | ||
| + | |From here on it will be shown how similar and not similar roots can be summed together in one expression. | ||
| + | |style="width:45%;text-align:right;"|ומכאן ולהבא רצוני להראותך כיצד שרשים שוים ו{{#annot:term|962|mEAx}}בלתי שוים{{#annotend:mEAx}} יכולי' לחברם יחד בקול אחד | ||
| + | |- | ||
| + | |Since many are those roots that cannot be summed in one expression, | ||
| + | |style="text-align:right;"|בהיות כי רבים הם אותם השרשי' אשר לא יתכן לחברם בקול אחד | ||
| + | |- | ||
| + | |and the nature of these roots, that cannot be summed together, is that when the numbers, by which the roots are denominated, are multiplied by each other, and that product does not have an expressible root, these are the roots that cannot be summed in one expression. | ||
| + | |style="text-align:right;"|וטבע אותם השרשי' אשר לא יתכן לחברם יחד בקול אחד הוא זה כי בהכפל המספרי' אשר נקראי' שרשי' להם זה בזה ואותו הכפל אין לו {{#annot:term|1075,2192|4Qky}}שרש מדובר{{#annotend:4Qky}} אלו השרשי' לא יתכן לחברם בקול אחד | ||
| + | |- | ||
| + | |Hence, these roots - which the multiplication of their numbers, by which they are denominated, by each other, yields a number that has an expressible root - can be summed in one expression, as will be demonstrated from here on. | ||
| + | |style="text-align:right;"|א"כ אותם השרשי' אשר בהכפל מספריהם אשר נקראו שרשי' להם זה בזה יעשה מספר שיהיה לו שרש מדובר יתכן לחברם בקול אחד כמו שאראך מכאן ולהבא | ||
| + | |- | ||
| + | |The addition method of roots with roots and roots with numbers | ||
| + | |style="text-align:right;"|בכאן יראה אופן {{#annot:term|154,1208|ZO9e}}חבור{{#annotend:ZO9e}} שרשי' עם שרשי' ושרשי' עם מספרי' או כאשר תרצה | ||
| + | |- | ||
| + | | | ||
| + | ==== <span style=color:Green>Addition of a Root with a Root</span> ==== | ||
| + | |||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:√3+√12|738|c9le}}<math>\scriptstyle\sqrt{3}+\sqrt{12}</math> | ||
| + | |style="text-align:right;"|<big>נניח</big> שרצית לחבר שרש ג' עם שרש י"ב{{#annotend:c9le}} | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{2\sdot\left(\sqrt{3}\sdot\sqrt{12}\right)=2\sdot\sqrt{36}=2\sdot6=12}}</math> | ||
| + | |style="text-align:right;"|הנך צריך לכפול שרש ג' בשרש י"ב ועושה שרש מל"ו<br> | ||
| + | ו{{#annot:term|795,1375|92E1}}תקח שרש{{#annotend:92E1}} זה הל"ו שהוא ו‫'<br> | ||
| + | ו{{#annot:term|785,1230|Ln1v}}תכפלהו{{#annotend:Ln1v}} ויהיה לך י"ב ותשמרם | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{3+12=15}}</math> | ||
| + | |style="text-align:right;"|אח"כ תחבר מספרי השרשי' שהם ג' וי"ב יחד ויהיו ט"ו | ||
| + | |- | ||
| + | | | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{3}+\sqrt{12}=\sqrt{12+15}=\sqrt{27}}}</math> | ||
| + | |style="text-align:right;"|וזה הט"ו תוסיף על י"ב ששמרת ויהיו כ"ז<br> | ||
| + | ושרש מכ"ז הוא {{#annot:term|388,1219|9yER}}נקבץ{{#annotend:9yER}} שרש ג' עם שרש מי"ב | ||
| + | |- | ||
| + | |It is also possible to add the roots mentioned in this way: | ||
| + | |style="text-align:right;"|<big>עוד</big> יתכן לחבר השרשי' הנזכרי' באופן זה | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{\sqrt{4\sdot\left(3\sdot12\right)}=\sqrt{4\sdot36}=\sqrt{144}=12}}</math> | ||
| + | |style="text-align:right;"|תכה מספרי השרשי' זה בזה דהיינו ג' בי"ב ויעלה ל"ו<br> | ||
| + | וזה הל"ו תכה בד' ויהיה לך קמ"ד<br> | ||
| + | {{#annot:term|795,1375|zzEO}}תקח שרשם{{#annotend:zzEO}} שהוא י"ב | ||
| + | |- | ||
| + | | | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{3}+\sqrt{12}=\sqrt{12+15}=\sqrt{27}}}</math> | ||
| + | |style="text-align:right;"|ותחברם עם מספרי השרשי' מקובצי' יחד דהינו על ט"ו ויהיה לך כ"ז<br> | ||
| + | ושרש זה הכ"ז הוא שני השרשי' מקובצים יחד כמו שאמרנו למעלה | ||
| + | |- | ||
| + | |For those that cannot be summed in one expression, the answer should be as they are, by expressing the one after the other: | ||
| + | |style="text-align:right;"|<big>ואותם</big> אשר לא יתכן לחברם בקול אחד ראוי לתשובתם כמו מה שהם באמור האחד אחר האחר | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:√6+√7|738|A3AW}}<math>\scriptstyle\sqrt{6}+\sqrt{7}</math> | ||
| + | |style="text-align:right;"|ונניח שרצית לחבר שרש ו' עם שרש ז‫'{{#annotend:A3AW}} | ||
| + | |- | ||
| + | | | ||
| + | ::One should answer and say "a root of 6 and a root of 7 with a root of 7 and a root of 6". | ||
| + | |style="text-align:right;"|אתה צריך להשיב ולומר שרש מו' ושרש מז' עם שרש ז' ושרש ו‫' | ||
| + | |- | ||
| + | |If one wishes to answer in another way, the answer would be more difficult. | ||
| + | |style="text-align:right;"|ואם רצונך להשיב לו באופן אחר יכבדו עליך יותר תשובותם | ||
| + | |- | ||
| + | |It is possible to answer in the aforesaid manner concerning the rule for those that are answered in one expression: | ||
| + | |style="text-align:right;"|ותוכל לענות ‫<ref>8v</ref>להם בזה האופן האמור למעלה בכלל אותם אשר יענו בקול אחד | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{\sqrt{4\sdot\left(6\sdot7\right)}=\sqrt{4\sdot42}=\sqrt{168}=12}}</math> | ||
| + | |style="text-align:right;"|והוא כי אתה צריך לכפול מספרי השרשי' זה בזה ועולה מ"ב<br> | ||
| + | וזה המ"ב תכה בד' ויהיה לך קס"ח | ||
| + | |- | ||
| + | | | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{6}+\sqrt{7}=\sqrt{\left(6+7\right)+\sqrt{168}}=\sqrt{13+\sqrt{168}}}}</math> | ||
| + | |style="text-align:right;"|ושרש קס"ח תחבר עם מספרי השרשי' הנקובי' בשם רצוני על שניהם שהוא י"ג ויהיה לך י"ג ושרש קס"ח<br> | ||
| + | ושרש זה הסך הוא שני השרשי' <s>או</s> האמורי' {{#annot:term|178,2083|nEt8}}מחוברי' יחד{{#annotend:nEt8}}<br> | ||
| + | א"כ תוכל לענות כי אלו שני השרשי' מחוברי' יחד הם שרש מנוסף י"ג עם שרש מקס"ח | ||
| + | |- | ||
| + | |Addition of a number and a root with a number and a root - adding the number to the number, then the roots to the roots, in the manner stated above. | ||
| + | |style="text-align:right;"|ואם יזדמן לך לחבר מספר ושרש עם מספר ושרש אתה צריך לחבר המספרי' עם המספרי' ואח"כ השרשי' עם השרשי' באופן האמו' למעלה | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | ==== <span style=color:Green>Subtraction of a Root from a Root</span> ==== | ||
| + | |||
| + | | | ||
| + | |- | ||
| + | |Know that as in the addition of the roots it was taught that summing two roots together is possible only for those, which when one of them is multiplied by the other, it yields a number that has an expressible root, likewise in the subtraction of the roots, it is possible to subtract the one from the other, so that the remainder will remain in one expression, only for those roots, which when one is multiplied by the other, it yields a number that has an expressible root. | ||
| + | |style="width:45%;text-align:right;"|<big>ודע</big> כי כמו שבחבור השרשי' נלמד כי לא יתכן החבור בקול אחד שני שרשי' יחד רק אותם אשר כשהוכה האחד באחר עושה מספר שיש לו שרש מדובר כמו כן ב{{#annot:term|155,1248|P7E1}}גרעון{{#annotend:P7E1}} השרשי' לא יתכן לגרוע האחד מהאחר <s>ושרש</s> וש{{#annot:term|184,1236|wr1s}}הנשאר{{#annotend:wr1s}} [ישאר]‫<ref>om.</ref> בקול אחד כי אם אותם השרשי' אשר כשהוכה אחד מהם באחר עושה מספר שיש לו <s>מס</s> שרש מדובר | ||
| + | |- | ||
| + | |As those that cannot be summed in one expression are stated as "a root of this plus a root of that", also those roots that cannot be subtracted in one expression can be answered as "a root of this minus a root of that". | ||
| + | |style="text-align:right;"|וכמו שאותם אשר לא יתכן לחברם בקול אחד יאמר שרש מכך ושרש מכך כן ג"כ אותם השרשי' אשר לא יתכן להוציאם בקול אחד נוכל לענות שרש מכך פחות שרש מכך | ||
| + | |- | ||
| + | |This is by stating the greater first, minus the smaller, as I will show you in the example below: | ||
| + | |style="text-align:right;"|וזה באמור הגדול תחלה פחות הקטון כמו שאראך לדמיון פה למטה | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:√12-√3|739|1zTf}}Suppose you wish to subtract a root of 3 from a root of 12. | ||
| + | :<math>\scriptstyle\sqrt{12}-\sqrt{3}</math> | ||
| + | |style="text-align:right;"|<big>נניח</big> שרצית לגרוע שרש ג' משרש י"ב{{#annotend:1zTf}} | ||
| + | |- | ||
| + | | | ||
| + | :You should apply the rule stated for addition that is to multiply a root of 3 by a root of 12, which yields a root of 36. Extract its expressible root, which is 6, and double it; it is 12. Keep it. | ||
| + | :<math>\scriptstyle{\color{blue}{2\sdot\left(\sqrt{3}\sdot\sqrt{12}\right)=2\sdot\sqrt{36}=2\sdot6=12}}</math> | ||
| + | |style="text-align:right;"|הנך צריך לפעול עם הכלל האמור בחבור בכמו שהוא להכות שרש ג' בשרש י"ב שעושה שרש ל"ו וקח שרשו המדובר שהוא ו' ו{{#annot:term|785,1230|zfVw}}כפלהו{{#annotend:zfVw}} ויהיה י"ב ושמרם | ||
| + | |- | ||
| + | | | ||
| + | :Then sum the numbers of the roots together, i.e. 3 with 12; it yields 15. | ||
| + | :<math>\scriptstyle{\color{blue}{3+12=15}}</math> | ||
| + | |style="text-align:right;"|ואח"כ תחבר מספרי השרשי' יחד דהיינו ג' עם י"ב ועושה ט"ו | ||
| + | |- | ||
| + | | | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{12}-\sqrt{3}=\sqrt{15-12}=\sqrt{3}}}</math> | ||
| + | |style="text-align:right;"|וכמו שבחבור השרשי' יחובר הי"ב {{#annot:term|960,1238|IWQm}}השמור{{#annotend:IWQm}} כן בגרעון השרשי' צריך לגרוע מזה הט"ו הי"ב השמור וישאר ג‫'<br> | ||
| + | ושרש זה הג' הוא {{#annot:term|184,1236|dhSG}}הנשאר מגרעון{{#annotend:dhSG}} שרש ג' משרש י"ב | ||
| + | |- | ||
| + | |The other way that is done in the addition, is done also in the subtraction, | ||
| + | |style="text-align:right;"|<big>עוד</big> באופן האחר שעושים בחבור כמו כן עושים במגרעת | ||
| + | |- | ||
| + | |except that as the root of the product by 4 is added to the numbers of the roots that are summed together, <math>\scriptstyle\sqrt{a}+\sqrt{b}=\sqrt{\left(a+b\right)+\sqrt{4\sdot\left(a\sdot b\right)}}</math> | ||
| + | |style="text-align:right;"|מלבד כי כמו ש<sup>י</sup>חובר השרש מהכפל שהוכפל בד' על מספרי השרשי' ש{{#annot:term|178,2083|Zy0c}}חוברו יחד{{#annotend:Zy0c}} | ||
| + | |- | ||
| + | |so the aforesaid root is subtracted from the stated numbers that are summed together, and the root of the remainder is the remainder of the subtraction of a certain root from another root. <math>\scriptstyle\sqrt{a}-\sqrt{b}=\sqrt{\left(a+b\right)-\sqrt{4\sdot\left(a\sdot b\right)}}</math> | ||
| + | |style="text-align:right;"|כן {{#annot:term|181,1365|E6D3}}יגרע ה{{#annotend:E6D3}}שרש האמור משני המספרי' האמורי' המחוברי' יחד ושרש הנשאר הוא {{#annot:term|184,2454|Lxgt}}השארית בגרעון{{#annotend:Lxgt}} שרש מה משרש אחר | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{\sqrt{4\sdot\left(3\sdot12\right)}=\sqrt{4\sdot36}=\sqrt{144}=12}}</math> | ||
| + | |style="text-align:right;"|באופן זה ברצותך להוציא שרש ג' משרש י"ב תכה ג' בי"ב עושה ל"ו<br> | ||
| + | וזה הל"ו תכה בד' ועולה קמ"ד<br> | ||
| + | ותקח שרשם והוא י"ב ושמור זה הי"ב | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{3+12=15}}</math> | ||
| + | |style="text-align:right;"|ועתה קבץ מספרי השרשי' אשר אתה בא להוציא האחד מן האחר שהוא ג' עם י"ב ועושה ט"ו | ||
| + | |- | ||
| + | | | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{12}-\sqrt{3}=\sqrt{15-12}=\sqrt{3}}}</math> | ||
| + | |style="text-align:right;"|ומזה הט"ו הוצא י"ב השמור וישאר ג‫'<br> | ||
| + | ושרש זה הג' הוא {{#annot:term|184,2454|DqCw}}השארית{{#annotend:DqCw}} הנשאר בהוציאנו שרש ג' משרש י"ב | ||
| + | |- | ||
| + | |Those roots that cannot be subtracted in one expression should be answered as they are, by stating the one, namely the greater, minus the other, namely the smaller: | ||
| + | |style="text-align:right;"|<big>ואותם</big> השרשים אשר לא יתכן להוציאם בקול אחד ראוי לענות בהם כפי מה שהם באמור האחד רצוני הגדול פחות האחר רצוני הקטון | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:√7-√6|739|VGtp}}<math>\scriptstyle\sqrt{7}-\sqrt{6}</math> | ||
| + | |style="text-align:right;"|ונניח שרצית להוציא שרש ו' משרש ז‫'{{#annotend:VGtp}} | ||
| + | |- | ||
| + | | | ||
| + | ::One should answer that a root of 7 minus a root of 6 remain. | ||
| + | |style="text-align:right;"|ראוי אתה לענות ‫<ref>9r</ref>שישאר שרש ז' פחות שרש ו‫' | ||
| + | |- | ||
| + | |If one wishes to answer in another way, it is possible to answer according to the rule for those that are answered in one expression, although the answer will consist of the said combination: | ||
| + | |style="text-align:right;"|ואם רצית לענות לו באופן אחר היית יכול <s>לענו</s> לענות לו כפי הכלל מהנענים בקול אחד <sup>גם</sup> כי התשובה תהיה מהרכבה חמורה | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{2\sdot\left(\sqrt{6}\sdot\sqrt{7}\right)=2\sdot\sqrt{42}=\sqrt{4\sdot42}=\sqrt{168}}}</math> | ||
| + | |style="text-align:right;"|בהיות כי אתה צריך לכפול שרש ו' בשרש ז' שעולה שרש מ"ב וזה המ"ב תכפול בב' שהוא <sup>ב</sup>שרש ד' ויהיה לך שרש קס"ח | ||
| + | |- | ||
| + | | | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{7}-\sqrt{6}=\sqrt{\left(7+6\right)-\sqrt{168}}=\sqrt{13-\sqrt{168}}}}</math> | ||
| + | |style="text-align:right;"|ושרש זה הקס"ח תוציא משני מספרי השרשי' מחוברים יחד שהוא י"ג וישאר י"ג פחות שרש קס"ח<br> | ||
| + | ושרש זה השארית הוא מה שישאר מהוצאת שרש ו' משרש ז‫'<br> | ||
| + | אם כן תהיה תשובתך כי השארית האמור הוא <sup>יהיה</sup> שרש מ{{#annot:term|155,1462|rrCQ}}הוצאת{{#annotend:rrCQ}} שרש מקס"ח מי"ג | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== <span style=color:Green>Addition of a Number and a Root with a Number and a Root</span> ==== | ||
| + | |||
| + | | | ||
| + | |- | ||
| + | |After demonstrating the addition and subtraction of one root from another, it will be shown how to add or subtract a number and a root from a number and a root, or a number and a root from a number minus a root, and a root minus a number with a root minus a number, or from a root minus a number, in many ways shown from here on. | ||
| + | |style="text-align:right;"|<big>אחרי</big> שהראיתיך לחבר ולהוציא שרש אחד מאחר רצוני להראותך כיצד נחבר או נוציא מספר ושרש ממספר ושרש או מספר ושרש ממספר פחות שרש ושרש פחות מספר <sup>עם</sup> <s>מ</s>שרש פחות מספר או משרש פחות מספר ובאופנים רבים אשר אתה מראה מכאן ולהבא | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:(4+√12)+(5+√3)|738|Jwfs}}<math>\scriptstyle\left(4+\sqrt{12}\right)+\left(5+\sqrt{3}\right)</math> | ||
| + | |style="text-align:right;"|<big>ונניח</big> שבקשת לחבר ד' ושרש י"ב עם ה' ושרש ג‫'{{#annotend:Jwfs}} | ||
| + | |- | ||
| + | |One should do as shown in the addition of roots, i.e. to sum the numbers with the numbers and the roots with the roots: | ||
| + | |style="text-align:right;"|הנך צריך לעשות כאשר הראנו לך תחת חבור השרשים דהיינו שאתה צריך לחבר המספרי' עם המספרי' והשרשי' עם השרשי‫' | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{4+5=9}}</math> | ||
| + | |style="text-align:right;"|ולכן תחבר ד' וה' ויהיו ט' ותשמרם | ||
| + | |- | ||
| + | | | ||
| + | ::summing the roots in the way of the rule stated for the addition | ||
| + | |style="text-align:right;"|אח"כ תחבר השרשי' באופן הכלל האמור בחבור | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{\sqrt{3}+\sqrt{12}=\sqrt{27}}}</math> | ||
| + | |style="text-align:right;"|דהיינו שרש ג' בשרש י"ב אשר עולה כ"ז | ||
| + | |- | ||
| + | | | ||
| + | :<math>\scriptstyle{\color{blue}{\left(4+\sqrt{12}\right)+\left(5+\sqrt{3}\right)=9+\sqrt{27}}}</math> | ||
| + | |style="text-align:right;"|אשר תחברהו אל {{#annot:term|960,1238|K3AP}}המספר השמור{{#annotend:K3AP}} שהוא ט' ו{{#annot:term|875,1240|pv3B}}יעלה ה{{#annotend:pv3B}}סך מאלו המספרי' והשרשי' מחוברי' יחד ט' ושרש כ"ז | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|בכאן יראה מספר ושרש עם מספר ושרש ובדברי' מה אחרים כאשר יראה בהמשך | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== <span style=color:Green>Addition of a Number and a Root with a Root minus a Number</span> ==== | ||
| + | |||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:(4+√3)+(√12-3)|738|MsOS}}<math>\scriptstyle\left(4+\sqrt{3}\right)+\left(\sqrt{12}-3\right)</math> | ||
| + | |style="text-align:right;"|<big>ואם</big> רצית לחבר ד' ושרש ג' עם שרש י"ב פחות ג‫'{{#annotend:MsOS}} | ||
| + | |- | ||
| + | |It should be done with the rule stated for addition above, | ||
| + | |style="text-align:right;"|צריך שתעשה עם הכלל האמור מהחבור למעלה | ||
| + | |- | ||
| + | |except that as for the addition of a number and a root with a root and a number the numbers should be summed together, | ||
| + | |style="text-align:right;"|מלבד שכמו שבעבור חבור מספ' ושרש עם שרש ומספר צריך לחבר המספרי' יחד | ||
| + | |- | ||
| + | |so in order to add a root and a number with a root minus a number, one number should be subtracted from the other: | ||
| + | |style="text-align:right;"|כמו כן לחבר שרש ומספר עם שרש פחות מספר צריך להוציא המספר האחד מהאחר | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:(4+√3)+(√12-3)|738|YJQX}}<math>\scriptstyle\left(4+\sqrt{3}\right)+\left(\sqrt{12}-3\right)</math> | ||
| + | |style="text-align:right;"|הדמיון לזה לחבר ד' ושרש ג' עם שרש י"ב פחות ג‫'{{#annotend:YJQX}} | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{\sqrt{3}+\sqrt{12}=\sqrt{27}}}</math> | ||
| + | |style="text-align:right;"|שצריך שנחבר שני השרשים יחד כאמור למעלה והנה {{#annot:term|388,1208|lRWw}}חבורם יחד{{#annotend:lRWw}} שרש מכ"ז ושמור | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{4-3=1}}</math> | ||
| + | |style="text-align:right;"|ועתה הוצא ג' מד' וישאר א‫'<br> | ||
| + | וזה אתה מוציא בעבור כי הנך אומר ג' פחות | ||
| + | |- | ||
| + | | | ||
| + | :<math>\scriptstyle{\color{blue}{\left(4+\sqrt{3}\right)+\left(\sqrt{12}-3\right)=\sqrt{27}+1}}</math> | ||
| + | |style="text-align:right;"|א"כ ד' ושרש ג' עם שרש י"ב פחות ג' עולה שרש מכ"ז וא' יותר | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ד' ושרש ג' בשרש י"ב פחות ג' עולה א' ושרש מכ"ז | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | *{{#annot:(4+√3)+(√12-2)|738|mDrF}}<math>\scriptstyle\left(4+\sqrt{3}\right)+\left(\sqrt{12}-2\right)</math> | ||
| + | |style="text-align:right;"|<big>עוד</big> אם יאמר לך אדם חבר ד' עם שרש ג' עם שרש י"ב פחות ב‫'{{#annotend:mDrF}} | ||
| + | |- | ||
| + | |One should subtract one root from the other, and one number from the other, since the name is denominated minus a root and minus a number, and the remainder will be the result of addition of a number minus a root with a root minus a number: | ||
| + | |style="text-align:right;"|דע כי צריך אתה להוציא השרש האחד מהאחר והמספר האחד מהאחר<br> | ||
| + | בעבור כי נקוב בשם פחות שרש ופחות מספר<br> | ||
| + | והנשאר יהיה העולה מחבור ‫<ref>9v</ref>מספר פחות שרש עם שרש פחות מספר | ||
| + | |- | ||
| + | | | ||
| + | ==== <span style=color:Green>Addition of a Number minus a Root with a Number minus a Root</span> ==== | ||
| + | |||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:(4-√3)+(√12-2)|738|N7Qh}}<math>\scriptstyle\left(4-\sqrt{3}\right)+\left(\sqrt{12}-2\right)</math> | ||
| + | |style="text-align:right;"|ומזה תקח המשל שיהיה ד' פחות שרש ג' עם שרש י"ב פחות ב‫'{{#annotend:N7Qh}} | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{4-2=2}}</math> | ||
| + | :<math>\scriptstyle{\color{blue}{\left(4-\sqrt{3}\right)+\left(\sqrt{12}-2\right)=\left(2-\sqrt{3}\right)+\sqrt{12}}}</math> | ||
| + | |style="text-align:right;"|תוציא ב' מד' וישאר ב' פחות שרש ג' החלק האחד והאחר ישאר אחר זה שרש מי"ב | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(2-\sqrt{3}\right)+\sqrt{12}=2+\left(\sqrt{12}-\sqrt{3}\right)}}</math> | ||
| + | |style="text-align:right;"|עתה תוציא שרש ג' מהחלק האחר וישאר החלק האחד ב' והאחר הוא <s>החלק</s> <sup>השרש</sup> האחד מי"ב פחות שרש ג‫' | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{\sqrt{12}-\sqrt{3}=\sqrt{3}}}</math> | ||
| + | |style="text-align:right;"|ולכן תוציא בדרך ה{{#annot:term|155,1462|pBiX}}הוצאה{{#annotend:pBiX}} האמור לפנים שרש ג' משרש י"ב וישאר שרש ג‫' | ||
| + | |- | ||
| + | | | ||
| + | :<math>\scriptstyle{\color{blue}{\left(4-\sqrt{3}\right)+\left(\sqrt{12}-2\right)=2+\sqrt{3}}}</math> | ||
| + | |style="text-align:right;"|וזה השרש תחבר עם ב' האמור קודם ויהיה לך ב' ושרש ג‫'<br> | ||
| + | וככה עולה לחבר ד' פחות שרש ג' עם שרש י"ב פחות ב‫' | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ד' פחות שרש ג' בשרש י"ב פחות ב' עולה ב' ושרש ג‫' | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== <span style=color:Green>Subtraction of a Number minus a Root from a Number</span> ==== | ||
| + | |||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:19-(10-√12)|739|UiBt}}<math>\scriptstyle19-\left(10-\sqrt{12}\right)</math> | ||
| + | |style="text-align:right;"|<big>ואם</big> רצית להוציא עשרה פחות שרש י"ב מי"ט{{#annotend:UiBt}} | ||
| + | |- | ||
| + | | | ||
| + | :<math>\scriptstyle{\color{blue}{19-\left(10-\sqrt{12}\right)=\left(19+\sqrt{12}\right)-10}}</math> | ||
| + | |style="text-align:right;"|הנך צריך לחבר השרש הפוחת מעשרה בחלק האחר דהיינו לשרש י"ב ויהיה לך י"ט ושרש י"ב | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{19-10=9}}</math> | ||
| + | |style="text-align:right;"|ועתה צריך אתה להוציא עשרה מי"ט וישאר ט‫' | ||
| + | |- | ||
| + | | | ||
| + | :<math>\scriptstyle{\color{blue}{19-\left(10-\sqrt{12}\right)=9+\sqrt{12}}}</math> | ||
| + | |style="text-align:right;"|א"כ להוציא עשרה פחות שרש י"ב מי"ט ישאר ט' ושרש י"ב | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== <span style=color:Green>Subtraction of a Number and a Root from a Number</span> ==== | ||
| + | |||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:16-(8+√50)|739|ouRF}}<math>\scriptstyle16-\left(8+\sqrt{50}\right)</math> | ||
| + | |style="text-align:right;"|<big>ואם</big> רצית להוציא ח' ושרש נ' מי"ו{{#annotend:ouRF}} | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{16-8=8}}</math> | ||
| + | |style="text-align:right;"|הנך צריך להוציא ח' מי"ו וישאר ח‫' | ||
| + | |- | ||
| + | | | ||
| + | :<math>\scriptstyle{\color{blue}{16-\left(8+\sqrt{50}\right)=8-\sqrt{50}}}</math> | ||
| + | |style="text-align:right;"|אח"כ הוציא שרש נ' מח' וישאר ח' פחות שרש נ‫' | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|מפני כי להוציא שרש מה ממספר מה לא יתכן לאמר יותר מדומה לזאת התשובה באמור המספר פחות השרש | ||
| + | |- | ||
| + | | | ||
| + | :<math>\scriptstyle{\color{blue}{16-\left(8+\sqrt{50}\right)=8-\sqrt{50}}}</math> | ||
| + | |style="text-align:right;"|א"כ להוציא ח' ושרש נ' מי"ו ישאר ח' פחות שרש נ' וכן נגמר ה{{#annot:term|469,1427|Vs9i}}מעשה{{#annotend:Vs9i}} | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== <span style=color:Green>Subtraction of a Number minus a Root from a Number</span> ==== | ||
| + | |||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:10-(24-√250)|739|K9xs}}<math>\scriptstyle10-\left(24-\sqrt{250}\right)</math> | ||
| + | |style="text-align:right;"|<big>עוד</big> אם בקשת להוציא כ"ד פחות שרש מר"נ מעשרה{{#annotend:K9xs}} | ||
| + | |- | ||
| + | | | ||
| + | :<math>\scriptstyle{\color{blue}{10-\left(24-\sqrt{250}\right)=\left(10+\sqrt{250}\right)-24}}</math> | ||
| + | |style="text-align:right;"|הנך צריך לחבר שרש ר"נ על עשרה ויהיה לך עשרה ושרש ר"נ | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{24-10=14}}</math> | ||
| + | |style="text-align:right;"|ועתה תוציא כ"ד מעשרה ושרש ר"נ יותר בזה האופן<br> | ||
| + | תוציא עשרה מכ"ד וישאר י"ד | ||
| + | |- | ||
| + | | | ||
| + | :<math>\scriptstyle{\color{blue}{10-\left(24-\sqrt{250}\right)=\sqrt{250}-14}}</math> | ||
| + | |style="text-align:right;"|וזה הי"ד הוא פוחת א"כ ישאר האחר שרש ר"נ פחות י"ד<br> | ||
| + | ו{{#annot:term|875,1231|0Vbm}}יצא לך כי{{#annotend:0Vbm}} בהוציאנו כ"ד פחות שרש ר"נ מעשרה ישאר שרש ר"נ פחות י"ד | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== <span style=color:Green>Subtraction of a Number minus a Root from a Number minus a Root</span> ==== | ||
| + | |||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:(13-√20)-(6-√5)|739|0TcW}}<math>\scriptstyle\left(13-\sqrt{20}\right)-\left(6-\sqrt{5}\right)</math> | ||
| + | |style="text-align:right;"|<big>עוד</big> אם יאמר לך תוציא ו' פחות שרש ה' מי"ג פחות שרש כ‫'{{#annotend:0TcW}} | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{13-6=7}}</math> | ||
| + | |style="text-align:right;"|אתה צריך להוציא ו' מי"ג וישאר ז‫' | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{\sqrt{20}-\sqrt{5}=\sqrt{5}}}</math> | ||
| + | |style="text-align:right;"|ואח"כ תוציא שרש ה' משרש כ' אשר כפי הכלל מהוצאת שרש אחד משרש אחר אשר הראנו לך קודם ישאר שרש ה‫' | ||
| + | |- | ||
| + | | | ||
| + | :<math>\scriptstyle{\color{blue}{\left(13-\sqrt{20}\right)-\left(6-\sqrt{5}\right)=7-\sqrt{5}}}</math> | ||
| + | |style="text-align:right;"|ויגרע ג"כ השרש האמור<br> | ||
| + | א"כ להוציא ו' פחות שרש ה' מי"ג פחות שרש כ' ישאר ז' פחות שרש ה‫' | ||
| + | |- | ||
| + | |Many other additions, as well as other subtractions, which were not written, not seen, and not taught, can occur. | ||
| + | |style="text-align:right;"|<big>ודע</big> כי הרבה {{#annot:term|154,1208|4FgY}}חבורי'{{#annotend:4FgY}} אחרים וכמו כן {{#annot:term|155,1462|IGX3}}הוצאות{{#annotend:IGX3}} אחרי' אפשר שיפלו אשר לא נכתבו גם לא נראו ולא נלמדו | ||
| + | |- | ||
| + | |Since the aforesaid rules of addition and subtraction of roots are enough for those stated above, and for any one that may occur, by observing each time what may be added or subtracted, as slightly seen in the stated rules. | ||
| + | |style="text-align:right;"|מפני כי הכללי' האמורים למעלה מהחבור וה{{#annot:term|155,1193|kkyF}}מגרעת{{#annotend:kkyF}} בשרשים הם מספיקים לאשר נאמרו למעלה ולכל אחד מאשר יוכלו להזדמן בהיותנו בכל פעם מתבוננים באשר אפשר להזדמן לחבר או להוציא כפי מה שהראית קצת בכללים האמורים | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== <span style=color:Green>Addition of Numerous Roots</span> ==== | ||
| + | |||
| + | | | ||
| + | |- | ||
| + | |If you wish to sum many types of roots together | ||
| + | |style="text-align:right;"|‫<ref>10r</ref><big>ואם</big> רצית לחבר מינים רבים משרשים יחד | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:√3+√6+√12+√24|738|Nydh}}<math>\scriptstyle\sqrt{3}+\sqrt{6}+\sqrt{12}+\sqrt{24}</math> | ||
| + | |style="text-align:right;"|נניח שרצונך לחבר שרש ג' עם שרש ו' ועם שרש י"ב ועם שרש כ"ד{{#annotend:Nydh}} | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|וכמו כן שרשי' אחרים אשר יזדמנו לך | ||
| + | |- | ||
| + | |The one should be multiplied by the other. | ||
| + | |style="text-align:right;"|הנך צריך לכפול האחד באחר | ||
| + | |- | ||
| + | |If the product has no root - the product of one of the terms by one of the other terms should be investigated. | ||
| + | |style="text-align:right;"|ואם אותו כפל לא יהיה לו שרש הנך צריך לחקור מכפול אחד מאותם החלקי' באחד מן החלקי' האחרי‫' | ||
| + | |- | ||
| + | |Then, what is discovered as possible to be summed, is summed in one expression | ||
| + | |style="text-align:right;"|ולחבר בקול אחד אשר תמצא שאפשר לחברם | ||
| + | |- | ||
| + | |and what is not, is answered in the manner stated before for the roots that cannot be summed in one expression | ||
| + | |style="text-align:right;"|ואשר לא תמצאם תענם באופן האמור קודם מהשרשי' אשר לא יתכן לחברם בקול אחד | ||
| + | |- | ||
| + | | | ||
| + | ::the first multiplied by the second: <math>\scriptstyle{\color{blue}{\sqrt{3}\sdot\sqrt{6}=\sqrt{18}}}</math> | ||
| + | |style="text-align:right;"|א"כ תכפול הראשון בשני דהינו שרש ג' בשרש ו' שעולה שרש י"ח | ||
| + | |- | ||
| + | | | ||
| + | ::this root is inexpressible | ||
| + | |style="text-align:right;"|וזה {{#annot:term|2370,2192|IRIo}}השרש אינו מדובר{{#annotend:IRIo}} | ||
| + | |- | ||
| + | | | ||
| + | ::These two roots cannot be summed in one expression | ||
| + | |style="text-align:right;"|שני אלו השרשי' לא יתכן לחברם בקול אחד | ||
| + | |- | ||
| + | | | ||
| + | ::[the first multiplied] by the third: <math>\scriptstyle{\color{blue}{\sqrt{3}\sdot\sqrt{12}=\sqrt{36}=6}}</math> | ||
| + | ::this root is expressible | ||
| + | |style="text-align:right;"|ולכן תנסה שרש ג' עם השרש השלישי שהוא שרש י"ב שעושה שרש ל"ו<br> | ||
| + | וזה השרש הוא מדבר והנה הוא ו‫' | ||
| + | |- | ||
| + | | | ||
| + | ::so, these two roots can be summed in one expression in the aforesaid manner | ||
| + | ::<math>\scriptstyle{\color{blue}{\sqrt{3}+\sqrt{12}=\sqrt{27}}}</math> | ||
| + | |style="text-align:right;"|א"כ אלו שני השרשי' אפשר לחברם בקול אחד באופן האמור למעלה והנה הוא יהיה שרש מכ"ז | ||
| + | |- | ||
| + | | | ||
| + | ::If it were impossible to sum these roots together in one expression, then the fourth should have been examined, as well as the second [multiplied] by the third and by the fourth | ||
| + | |style="text-align:right;"|ואם אלו השרשי' לא היה אפשר לחברם יחד בקול אחד אז היית מנסה ברביעי וכמו כן השני בשלישי וברביעי | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|וכן בנסות וכָפוֹל האחד באחר עד כלותך לנסות כל אחד מהם או מאחרים שיזדמנו | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{\sqrt{6}+\sqrt{24}=\sqrt{54}}}</math> | ||
| + | |style="text-align:right;"|ודע כי השני אפשר לחבר בקול אחד עם הרביעי ויהיה שרש מנ"ד כאשר חוברו יחד | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ויהיה לך כי אלו ד' השרשי' יהיו כאשר חוברו יחד בשני הסכי' רצוני הראשון בשלישי שהוא יהיה שרש מכ"ז והשני ברביעי שהוא יהיה שרש מנ"ד | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{3}+\sqrt{6}+\sqrt{12}+\sqrt{24}=\left(\sqrt{3}+\sqrt{12}\right)+\left(\sqrt{6}+\sqrt{24}\right)=\sqrt{27}+\sqrt{54}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ובזה האופן בעצמו ראוי שתבקש בכפול האחד על האחר ברצותך להוציא שרש אחד מב' שרשי' או ב' שרשי' מב' שרשים או <sup>ב'</sup> מג' וכדומה לזה בכל אופן מכמויות שרשים שיגיעו או שאפשר שיגיעו | ||
| + | |- | ||
| + | | | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{3}+\sqrt{6}+\sqrt{12}+\sqrt{24}=\sqrt{27}+\sqrt{54}}}</math> | ||
| + | |style="text-align:right;"|א"כ לחבר שרש ג' ושרש ו' ושרש י"ב ושרש כ"ד יעלה שרש כ"ז ושרש מנ"ד | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | === <span style=color:Green>Division of Roots</span> === | ||
| + | |||
| + | | | ||
| + | |- | ||
| + | |After you have seen the teaching of multiplication, addition and subtraction of roots, it remains for you to see the teaching of the division of roots, I mean one root by another root, a number by a root, a root by a number, a number and a root by a number, a number by a root and a number, a root by a number and a root, a number and a root by a number and a root, a root and a number by a number minus a root, and any manner that may occur | ||
| + | |style="width:45%;text-align:right;"|<big>אחר</big> אשר ראית התלמדות הכפל והחבור והמגרעת בשרשים נשאר לך לראות התלמדות ה{{#annot:term|157,1223|MLlZ}}חלוק{{#annotend:MLlZ}} בשרשים רצוני שרש אחד בשרש אחר או מספר בשרש או שרש במספר או מספר ושרש במספר או מספר בשרש ומספר או שרש במספר ושרש או מספר ושרש במספר ושרש או שרש ומספר במספר פחות שרש ובכל אופן שיוכל להגיע | ||
| + | |- | ||
| + | |Know that in the division of a certain root by another root you should divide the number of the first root by the number of the other, and the root of the quotient is the result of the division. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\sqrt{a}\div\sqrt{b}=\sqrt{\frac{a}{b}}}}</math> | ||
| + | |style="text-align:right;"|<big>ודע</big> כי בחלוק שרש <sup>מה</sup> בשרש אחר הנך צריך לחלוק המספר מהשרש האחד במספר מהשרש האחר ושרש מ{{#annot:term|783,2086|b3N0}}המספר המגיע מן החלוקה{{#annotend:b3N0}} הוא {{#annot:term|783,1223|lP6v}}החלוק{{#annotend:lP6v}} | ||
| + | |- | ||
| + | |Since the [root of the] quotient of one number by another number is the same as the expression of the root of the one from the expression of the root of the other | ||
| + | |style="text-align:right;"|מפני כי כן הוא חלק מספר אחד ממספר אחר כקול שרש <sup>ה</sup>אחד מקול שרש <sup>ה</sup>אחר | ||
| + | |- | ||
| + | |Here is the example of this: | ||
| + | |style="text-align:right;"|וזה הוא דמיונו | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:√4÷√9|740|i3Y0}}Suppose you wish to divide a root of 4 by a root of 9. | ||
| + | :<math>\scriptstyle\sqrt{4}\div\sqrt{9}</math> | ||
| + | |style="text-align:right;"|נניח שרצית לחלק שרש ד' בשרש ט‫'{{#annotend:i3Y0}} | ||
| + | |- | ||
| + | | | ||
| + | :You should divide 4 by 9; the result is 4-ninths. | ||
| + | :<math>\scriptstyle{\color{blue}{4\div9=\frac{4}{9}}}</math> | ||
| + | |style="text-align:right;"|הנך צריך לחלק ד' בט' שעולה ד' תשיעיות | ||
| + | |- | ||
| + | | | ||
| + | :The root of these 4-ninths is the quotient resulting from the division of a root of 4 by a root of 9 and the expression of its root is 2-thirds. | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{4}\div\sqrt{9}=\sqrt{\frac{4}{9}}=\frac{2}{3}}}</math> | ||
| + | |style="text-align:right;"|ושרש זה אלו הד' תשיעיות הוא החלוק הבא לחלוק שרש ד' בשרש ט' אשר זה הנגון או אמור הקול מד' תשיעיות המהות משרשו הוא ב' שלישיות | ||
| + | |- | ||
| + | | | ||
| + | :So, the result of division of a root of 4 by a root of 9 is a root of 4-ninths, which is 2-thirds. | ||
| + | |style="text-align:right;"|אם כן לחלוק שרש ד' בשרש ט' עולה שרש מד' תשיעיות שהוא ב' שלישיות | ||
| + | |- | ||
| + | | | ||
| + | ==== <span style=color:Green>Division of a Number by a Root</span> ==== | ||
| + | | | ||
| + | |- | ||
| + | |If you wish to divide a number by a root: | ||
| + | |style="text-align:right;"|‫<ref>10v</ref><big>ואם</big> רצית לחלק מספר בשרש | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:4÷√9|740|o4Lu}}Suppose you wish to divide 4 by a root of 9. | ||
| + | :<math>\scriptstyle4\div\sqrt{9}</math> | ||
| + | |style="text-align:right;"|ונניח שרצית לחלק ד' בשרש ט‫'{{#annotend:o4Lu}} | ||
| + | |- | ||
| + | | | ||
| + | :First, you should convert 4 into a root; you get a root of 16. | ||
| + | |style="text-align:right;"|הנך צריך ראשונה להשיב ד' אל שרש שיהיה לך שרש י"ו | ||
| + | |- | ||
| + | | | ||
| + | :Now, divide 16 by 9; the result is 1 and 7-ninths. | ||
| + | |style="text-align:right;"|ועתה תחלק י"ו על ט' ו{{#annot:term|875,1575|aTkY}}יגיע{{#annotend:aTkY}} א' וז' תשיעיות | ||
| + | |- | ||
| + | | | ||
| + | :The root of 1 and 7-ninths is the quotient resulting from the division of a root of 16, which is the stated 4, by a root of 9. The root of this quotient is 1 and a third. | ||
| + | :<math>\scriptstyle{\color{blue}{4\div\sqrt{9}=\sqrt{16}\div\sqrt{9}=\sqrt{\frac{16}{9}}=\sqrt{1+\frac{7}{9}}=1+\frac{1}{3}}}</math> | ||
| + | |style="text-align:right;"|ושרש א' וז' תשיעיות הוא {{#annot:term|783,1223|gR5o}}החלוק הבא בַחלוק{{#annotend:gR5o}} שרש י"ו שהוא ד' האמור לחלק מספר בשרש ט' ושרש זה החלוק הוא א' ו<s>ב'</s><sup>א'</sup> שליש | ||
| + | |- | ||
| + | | | ||
| + | :So, the result of division of 4 by a root of 9 is [a root of] 1 and 7-ninths, which is 1 and a third. | ||
| + | |style="text-align:right;"|א"כ לחלוק ד' בשרש ט' יגיע [שרש]‫<ref>marg.</ref> א' וז' תשיעיות שהוא א' ושליש ונשלם | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== <span style=color:Green>Division of a Number by a Root and a Number</span> ==== | ||
| + | | | ||
| + | |- | ||
| + | |If you wish to divide a number [by] a root [and] a number: | ||
| + | |style="width:45%;text-align:right;"|<big>ואם</big> רצית לחלוק מספר ושרש במספר | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:8÷(3+√4)|740|qqDu}}Suppose you wish to divide 8 by 3 plus a root of 4. | ||
| + | :<math>\scriptstyle8\div\left(3+\sqrt{4}\right)</math> | ||
| + | |style="text-align:right;"|נניח שרצית לחלוק ח' בג' ושרש ד‫'{{#annotend:qqDu}} | ||
| + | |- | ||
| + | | | ||
| + | :You should multiply 3 plus a root of 4 by 4 minus a root of 4; the result is 5. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(3+\sqrt{4}\right)\sdot\left(3-\sqrt{4}\right)=5}}</math> | ||
| + | |style="text-align:right;"|הנך צריך לכפול ג' ושרש ד' בג' פחות שרש ד' ויעלה ה‫' | ||
| + | |- | ||
| + | | | ||
| + | :Therefore, when dividing 5 by 3 plus a root of 4, you get 3 minus a root of 4. | ||
| + | :<math>\scriptstyle{\color{blue}{5\div\left(3+\sqrt{4}\right)=3-\sqrt{4}}}</math> | ||
| + | |style="text-align:right;"|א"כ לחלוק ה' בג' ושרש ד' יעלה לך ג' פחות שרש ד‫' | ||
| + | |- | ||
| + | |Since, for every number that is multiplied by another, when the product is divided by that number, the result is the other number by which it was multiplied. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\left(A\sdot B\right)\div A=B}}</math> | ||
| + | |style="text-align:right;"|מפני כי כל מספר שיוכה במספר אחר הכפל המגיע <s>הנחלק</s><sup>כשנחלק</sup> באותו מספר יגיע המספר האחר אשר הוכה בו | ||
| + | |- | ||
| + | | | ||
| + | :So, when dividing 5 by 3 plus a root of 4, the result is 3 minus a root of 4. | ||
| + | :<math>\scriptstyle{\color{blue}{5\div\left(3+\sqrt{4}\right)=3-\sqrt{4}}}</math> | ||
| + | |style="text-align:right;"|ולכן בחלוק ה' בג' ושרש ד' יגיע מזה ג' פחות שרש ד‫' | ||
| + | |- | ||
| + | | | ||
| + | :And, when dividing 5 by 3 minus a root of 4, the result is the other part, which is 3 plus a root of 4. | ||
| + | :<math>\scriptstyle{\color{blue}{5\div\left(3-\sqrt{4}\right)=3+\sqrt{4}}}</math> | ||
| + | |style="text-align:right;"|ובחלוק ה' בג' פחות שרש ד' יגיע מזה החלק האחר שהוא שרש ג' ושרש ד‫' | ||
| + | |- | ||
| + | | | ||
| + | :Hence, it is said that 5 is the denominator. | ||
| + | |style="text-align:right;"|ולכן נאמר כי זה הה' יהיה {{#annot:term|604,1228|PG8h}}המחלק{{#annotend:PG8h}} | ||
| + | |- | ||
| + | | | ||
| + | :We set this division in rule three and say: if when 5 is divided by 3 plus a root of 4, the result is 3 minus a root of 4, how much is obtained from 8 that we wish to divide? | ||
| + | :<math>\scriptstyle{\color{blue}{\left[5\div\left(3+\sqrt{4}\right)\right]:\left(3-\sqrt{4}\right)=\left[8\div\left(3+\sqrt{4}\right)\right]:a}}</math> | ||
| + | |style="text-align:right;"|ונשים זאת החלוקה בכלל הג' ונאמר אם מה‫' {{#annot:term|784,1259|FUXT}}בחלקו ב{{#annotend:FUXT}}ג' ושרש ד' יגיע ג' פחות שרש ד' כמה יגיע מח' אשר רצינו לחלקו | ||
| + | |- | ||
| + | | | ||
| + | :I.e. if 3 minus a root of 4 results from 5, how much will result from 8? | ||
| + | :<math>\scriptstyle{\color{blue}{5:\left(3-\sqrt{4}\right)=8:a}}</math> | ||
| + | |style="text-align:right;"|דהינו אם מה' יגיע ג' פחות שרש ד' כמה ראוי להגיע מח‫' | ||
| + | |- | ||
| + | | | ||
| + | :Multiply 3 minus a root of 4 by 8; the result is 24 minus a root of 256. | ||
| + | |style="text-align:right;"|תכפול ג' פחות שרש ד' <s>ש</s> בח' שעולה כ"ד פחות שרש רנ"ו | ||
| + | |- | ||
| + | | | ||
| + | :Divide this product by 5; the result is 4 and 4-fifths for the number. | ||
| + | |style="text-align:right;"|<s>וזאת הח</s> וזה הכפל תחלק בה' ויגיע ד' וד' חמישיות בעד המספר | ||
| + | |- | ||
| + | | | ||
| + | :Now, it remains to divide a root of 256 by 5, i.e. by a root of 25; the result is a root of ten plus 6 parts of 25. | ||
| + | |style="text-align:right;"|עתה נשאר לחלק שרש רנ"ו בה' דהינו בשרש כ"ה שיבא שרש עשרה וו' חלקי' מכ"ה | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{8\div\left(3+\sqrt{4}\right)=\frac{\left(3-\sqrt{4}\right)\sdot8}{5}=\frac{24-\sqrt{256}}{5}=\left(4+\frac{4}{5}\right)-\frac{\sqrt{256}}{\sqrt{25}}=\left(4+\frac{4}{5}\right)-\sqrt{10+\frac{6}{25}}}}</math> | ||
| + | |- | ||
| + | |Since in the division of roots by numbers, the number should be converted to roots, as it is converted in the division of numbers by roots. | ||
| + | |style="text-align:right;"|מפני כי ב{{#annot:term|157,1221|iGwr}}חלוקת{{#annotend:iGwr}} שרשי' במספרים צריך להשיב המספר אל שרשי' כמו ש{{#annot:term|1071,2559|k75H}}יושב{{#annotend:k75H}} בחלוקת מספרי' בשרשי‫' | ||
| + | |- | ||
| + | | | ||
| + | :Therefore, when dividing 8 by 3 and a root of 4, the result is 4 and 4-fifths minus a root of ten and 6 parts of 25. | ||
| + | :<math>\scriptstyle{\color{blue}{8\div\left(3+\sqrt{4}\right)=\left(4+\frac{4}{5}\right)-\sqrt{10+\frac{6}{25}}}}</math> | ||
| + | |style="text-align:right;"|ולכן בחלוק <s>ג'</s> ח' בג' ושרש ד' יגיע ד' וד' חמשים פחות שרש עשרה וו' חלקי' מכ"ה | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== <span style=color:Green>Division of a Number and a Root by a Number</span> ==== | ||
| + | | | ||
| + | |- | ||
| + | |If you wish to divide a number and a root by a number: | ||
| + | |style="width:45%;text-align:right;"|<big>ואם</big> רצית לחלק מספר ושרש במספר ושרש | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:(5+√16)÷3|740|snlR}}Suppose you wish to divide 5 plus a root of 16 by 3. | ||
| + | :<math>\scriptstyle\left(5+\sqrt{16}\right)\div3</math> | ||
| + | |style="text-align:right;"|נניח שרצית לחלק ה' ושרש י"ו בג‫'{{#annotend:snlR}} | ||
| + | |- | ||
| + | | | ||
| + | :First, you should divide the number by the number, i.e. 5 by 3; the result is 1 and 2-thirds. keep it. | ||
| + | :<math>\scriptstyle{\color{blue}{5\div3=1+\frac{2}{3}}}</math> | ||
| + | |style="text-align:right;"|אתה צריך ראשונה לחלק המספר במספר דהיינו ה' בג' שיגיע א' וב' שלישים ושמרם | ||
| + | |- | ||
| + | | | ||
| + | :Then, convert the divisor 3 into roots; you have a root of 9. | ||
| + | |style="text-align:right;"|אח"כ השב ג' המחלק אל שרשים ויהיה לך שרש ט‫' | ||
| + | |- | ||
| + | | | ||
| + | :Divide a root of 16 by a root of 9; the result is 1 and 7-ninths. | ||
| + | |style="text-align:right;"|אח"כ חלק שרש מי"ו בשרש ט' ויגי<sup>ע</sup> שרש מא' וז' תשיעיות | ||
| + | |- | ||
| + | | | ||
| + | :I.e. a root of the quotient of 16 by 9. | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{16}\div3=\sqrt{16}\div\sqrt{9}=\sqrt{\frac{16}{9}}=\sqrt{1+\frac{7}{9}}}}</math> | ||
| + | |style="text-align:right;"|דהיינו שרש מכמו שיגיע בחלוקת י"ו בט‫' | ||
| + | |- | ||
| + | | | ||
| + | :So, the result of division of 5 and a root of 16 by 3 is 1 and 2-thirds plus a root of 1 and 7-ninths. The total quotient is 3, because a root of 1 and 7-ninths is 1 and a third. | ||
| + | |style="text-align:right;"|א"כ לחלוק ה' ושרש י"ו בג' יעלה א' וב' שלישים ושרש א' וז' תשיעיות אשר זאת החלוקה בכלל תהיה ג' מפני כי שרש א' וז' תשיעיות הוא א' ושליש | ||
| + | |- | ||
| + | | | ||
| + | :The result of division of 5 and a root of 16 by 3 is 1 and 2-thirds plus a root of 1 and 7-ninths, which is 3 integers. | ||
| + | |style="text-align:right;"|לחלוק ה' ושרש י"ו בג' יגיע א' וב' שלישי ושרש מא' וז' תשיעיו' שהם ג' {{#annot:term|20,1268|A11m}}שלמי‫'{{#annotend:A11m}} | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{\left(5+\sqrt{16}\right)\div3=\left(1+\frac{2}{3}\right)+\sqrt{1+\frac{7}{9}}=\left(1+\frac{2}{3}\right)+\left(1+\frac{1}{3}\right)=3}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :The dividend is 9. | ||
| + | :<math>\scriptstyle{\color{blue}{5+\sqrt{16}=9}}</math> | ||
| + | |style="text-align:right;"|ו{{#annot:term|605,1226|9YPR}}המספר הנחלק{{#annotend:9YPR}} הוא ט‫' | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== <span style=color:Green>Division of a Number by a Number minus a Root</span> ==== | ||
| + | | | ||
| + | |- | ||
| + | |If you wish to divide a number by a number minus a root. | ||
| + | |style="text-align:right;"|<big>ואם</big> רצית לחלק מספר במספר פחות שרש | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:20÷(4-√9)|740|dg3U}}Suppose you wish to divide 20 by 4 minus a root of 9. | ||
| + | :<math>\scriptstyle20\div\left(4-\sqrt{9}\right)</math> | ||
| + | |style="text-align:right;"|נניח שבקשת לחלק כ' בד' פחות שרש ט‫'{{#annotend:dg3U}} | ||
| + | |- | ||
| + | |You should do it according to the aforesaid rule of the division of a number by a root and a number, except that when [you divide by a number and a root you should multiply] by a number minus a root, whereas in the division by a number minus a root, you should multiply by the inverse, i.e. by a number plus a root. | ||
| + | |style="width:45%;text-align:right;"|הנך צריך לעשות עם הכלל האמור לפנים מחלוקת מספר בשרש ומספר מלבד שאתה אם תכפול מספר ושרש במספר פחות שרש כן בחלוקת במספר פחות שרש צריך לכפול להפך דהיינו במספר ושרש | ||
| + | |- | ||
| + | |This is done in order that the divisor would be an integer. | ||
| + | |style="text-align:right;"|אשר זה יעשה בסבת שהמחלק יהיה {{#annot:term|20,1268|xzGs}}מספר שלם{{#annotend:xzGs}} | ||
| + | |- | ||
| + | |This teaching is found in the multiplication of roots, when a number plus a root is multiplied by the same number minus the same root [<math>\scriptstyle{\color{OliveGreen}{\left(a+\sqrt{b}\right)\times\left(a-\sqrt{b}\right)}}</math>], or a root plus a number by a root minus a number, when the numbers are equal to one another, meaning the subtractive to the additive, and the roots to each other [<math>\scriptstyle{\color{OliveGreen}{\left(\sqrt{a}+b\right)\times\left(\sqrt{a}-b\right)}}</math>], because the said products always yield integers | ||
| + | |style="text-align:right;"|וזה ההתלמדות ‫<ref>11r</ref>נמצא ב{{#annot:term|156,1253|G42r}}כפילת ה{{#annotend:G42r}}שרשי' כאשר הזדמן שיכפל מספר ושרש בכך מספר פחות <sup>שרש</sup> כך <s>מספ או מספר</s> או שרש ומספר בשרש פחות מספר בהיות המספרי' שוים זה לזה רצוני את הגורע ל{{#annot:term|788,2537|AGuK}}מרבה{{#annotend:AGuK}} והשרשי' זה לזה כי לעולם הכפלי' האמורי' עושים {{#annot:term|20,1268|Cn6i}}מספרי' שלמים{{#annotend:Cn6i}} | ||
| + | |- | ||
| + | | | ||
| + | :So, multiply 4 minus a root of 9 by 4 plus a root of 9; it yields 7, which is the denominator for the reason stated above concerning the division by a number and a root. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(4-\sqrt{9}\right)\sdot\left(4+\sqrt{9}\right)}}</math> = 7 | ||
| + | |style="text-align:right;"|ולכן תכה ד' פחות שרש ט' בד' ושרש ט' ועושה ז' שהוא המחלק לסבה האמורה קודם בחלוקת בעד מספר ושרש | ||
| + | |- | ||
| + | | | ||
| + | :Therefore, we say: if 7 yields 4 and a root of 9, how much does 20 that we wish to divide yield? | ||
| + | :<math>\scriptstyle{\color{blue}{7:\left(4+\sqrt{9}\right)=20:a}}</math> | ||
| + | |style="text-align:right;"|א"כ נאמר אנחנו אם מז' יגיע ד' ושרש ט' כמה ראוי להגיע מכ' שהוא אשר רצינו לחלק | ||
| + | |- | ||
| + | | | ||
| + | :Multiply 4 and a root of 9 by 20; the result is 80 and a root of 3600. | ||
| + | |style="text-align:right;"|תכה ד' ושרש ט' בכ' ועולה פ' ושרש מג' אלפי' ת"ר | ||
| + | |- | ||
| + | | | ||
| + | :Divide it by 7; the result is 11 and 3-sevenths plus a root of 73 and 23 parts of 49. | ||
| + | |style="text-align:right;"|וחלק בז' שיגיע י"א וג' שביעיו' ושרש ע"ג וכ"ג חלקי' ממ"ט | ||
| + | |- | ||
| + | | | ||
| + | :So, the result of division of 20 by 4 minus a root of 9 is 11 and 3-sevenths plus a root of 73 and 23 parts of 49. | ||
| + | |style="text-align:right;"|א"כ לחלוק כ' בד' פחות שרש ט' יגיע י"א וג' שביעיו' ושרש ע"ג וכ"ג חלקים ממ"ט | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{20\div\left(4-\sqrt{9}\right)=\frac{\left(4+\sqrt{9}\right)\sdot20}{7}=\frac{80+\sqrt{3600}}{7}=\left(11+\frac{3}{7}\right)+\sqrt{73+\frac{23}{49}}}}</math> | ||
| + | |- | ||
| + | |Know that when any of these divisions occur, or a division by a root minus a number, you should continue according to the said rule, to multiply always by the inverse of the denominator, as seen in the example. | ||
| + | |style="text-align:right;"|<big>דע</big> כי כאשר יזדמן לך איזה מאלו ה{{#annot:term|157,1223|LTmk}}חלוקי'{{#annotend:LTmk}} או לחלוק בשרש פחות מספר תהיה ממשיך הענין בכלל האמור לכפול לעולם <s>בנ</s> כמספר המתנגד ב{{#annot:term|571,2269|SjG1}}איכות{{#annotend:SjG1}} כפי מה שהראית במשל | ||
| + | |- | ||
| + | |Know also that when you divide a certain number [or] a root by a certain root and a number, such that the product of the added later by itself is greater than the product of the former by itself, then the addition, meaning the divisor, should be reversed, by placing the one whose product by itself yields more always first. | ||
| + | |style="text-align:right;"|<big>עוד</big> דע כי כאשר יזדמן לך לחלוק מספר מה ושרש בשרש מה ומספר שאשר {{#annot:term|178,2083|P8Kr}}יחובר ל{{#annotend:P8Kr}}אשר יהיה נקוב ראשונה בהכפל בעצמו יעשה יותר מאשר נקוב ראשונה בהכפל בעצמו צריך להפך ה{{#annot:term|154|Jt7q}}דיציאוני{{#annotend:Jt7q}} רצוני החלוק ולשים לעולם העושה יותר בהכפל בעצמו קודם | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:19÷(2+√16)|740|6lxt}}Example: suppose you wish to divide 19 by 2 plus a root of 16. | ||
| + | :<math>\scriptstyle19\div\left(2+\sqrt{16}\right)</math> | ||
| + | |style="text-align:right;"|והמשל בזה נניח שרצית לחלק י"ט בב' ושרש י"ו{{#annotend:6lxt}} | ||
| + | |- | ||
| + | | | ||
| + | :According to the aforementioned rule, you should multiply 2 plus a root of 16 by 2 minus a root of 16. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(2+\sqrt{16}\right)\sdot\left(2-\sqrt{16}\right)}}</math> | ||
| + | |style="text-align:right;"|מן הכלל האמור הנך צריך לכפול ב' ושרש י"ו בב' פחות שרש י"ו | ||
| + | |- | ||
| + | | | ||
| + | :But, this multiplication is impossible, because a root of 16 is greater than 2, and we cannot subtract the greater from the smaller. | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{16}>2}}</math> | ||
| + | |style="text-align:right;"|אשר הכפל הזה לא יתכן וסבת זה היא כי שרש י"ו הוא יותר מב' והיותר לא נוכל להוציאו מהפחות | ||
| + | |- | ||
| + | | | ||
| + | :We can see it clearly, because the multiplication of a root of 16 by itself yields 16. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(\sqrt{16}\right)^2=16}}</math> | ||
| + | |style="text-align:right;"|וזה נוכל לראותו בפרהסיא כי בהכפל שרש מי"ו בעצמו עושה י"ו | ||
| + | |- | ||
| + | | | ||
| + | :The multiplication of 2 by itself yields 4. | ||
| + | :<math>\scriptstyle{\color{blue}{2^2=4}}</math> | ||
| + | |style="text-align:right;"|וב' בהכפל בעצמו עושה ד‫' | ||
| + | |- | ||
| + | | | ||
| + | :So, a root of 16 is greater than 2, which is a root of 4. | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{16}>\sqrt{4}=2}}</math> | ||
| + | |style="text-align:right;"|א"כ שרש י"ו הוא יותר מב' שהוא שרש ד‫' | ||
| + | |- | ||
| + | | | ||
| + | :As we cannot subtract 16 from 4, because it is greater than 4, so it is impossible to subtract a root of 16 from a root of 4. | ||
| + | :<math>\scriptstyle{\color{blue}{16>4\longrightarrow4-16=\varnothing\longrightarrow\sqrt{4}-\sqrt{16}=\varnothing}}</math> | ||
| + | |style="text-align:right;"|וכמו שי"ו לא יתכן להוציאו מד' מפני שהוא יותר מד' כמו כן שרש י"ו לא יתכן להוציאו משרש ד‫' | ||
| + | |- | ||
| + | | | ||
| + | :Therefore, when we wish to divide 19 by 2 and a root of 16, we reverse the divisor and say: by a root of 16 plus 2. | ||
| + | :<math>\scriptstyle{\color{blue}{19\div\left(2+\sqrt{16}\right)=19\div\left(\sqrt{16}+2\right)}}</math> | ||
| + | |style="text-align:right;"|ולכן ברצותנו לחלק י"ט בב' ושרש י"ו או מהפכי' החלוק ונאמר בשרש י"ו וב‫' | ||
| + | |- | ||
| + | | | ||
| + | :We do this in order to multiply a root of 16 plus 2 by a root of 16 minus 2, which we can do properly and the result is 12. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(\sqrt{16}+2\right)\sdot\left(\sqrt{16}-2\right)=12}}</math> | ||
| + | |style="text-align:right;"|וזה נעשה אנחנו כדי לכפול שרש י"ו וב' בשרש י"ו פחות ב' וזה בטוב נוכל לעשותו ועולה י"ב | ||
| + | |- | ||
| + | | | ||
| + | :We say by the rule of three: if 12 yields a root of 16 minus 2, how much does 19 yield? | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{12:\left(\sqrt{16}-2\right)=19:a}}</math> | ||
| + | |style="text-align:right;"|א"כ נאמר עם כלל הג' אם מי"ב יגיע שרש י"ו פחות ב' כמה יגיע מי"ט | ||
| + | |- | ||
| + | | | ||
| + | :Multiply a root of 16 minus 2 by 19; the result is a root of 5776 minus 38. | ||
| + | |style="text-align:right;"|תכפול שרש י"ו פחות ב' בי"ט ועולה שרש מה' אלפי' ותשע"ו פחות ל"ח | ||
| + | |- | ||
| + | | | ||
| + | :Divide it by 12; the result is a root of 40 and a ninth minus three and a sixth. | ||
| + | |style="text-align:right;"|ותחלקם בי"ב ויגיע שרש ממ' ותשיעית פחות שלשה ושתות | ||
| + | |- | ||
| + | | | ||
| + | :So, the result of division of 19 by 2 and a root of 16 is a root of 40 and a ninth minus 3 and a sixth. | ||
| + | |style="text-align:right;"|א"כ לחלוק י"ט בב' ושרש י"ו יגיע שרש מ' ותשיעי' פחות ג' ושתות | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{19\div\left(2+\sqrt{16}\right)=\frac{\left(\sqrt{16}-2\right)\sdot19}{12}=\frac{\sqrt{5776}-38}{12}=\sqrt{40+\frac{1}{9}}-\left(3+\frac{1}{6}\right)}}</math> | ||
| + | |- | ||
| + | |Knoe that when you have a number and a root [or] two equal roots, meaning that the root that you add to the number is as great as the number, or that there are two roots that are equal to one another, and one intend to divide by the sum of both, we cannot answer or deal with them by one of the said manners. | ||
| + | |style="text-align:right;"|<big>ודע</big> כי אם יזדמן לך מספר אחד ושרש או אחד וב' שרשי' שוים רצוני כי השרש שתחבר עם המספר יהיה גדול כמו המספר או שיהיו ב' שרשים שוים האחד לאחר <s>וצריך</s> <sup>ואם בא</sup> לחלק שניהם מחוברי' לא נוכל לענות או להתעסק בם באחד מהאופני' האמורי‫' | ||
| + | |- | ||
| + | |The reason for this is that when we wish to multiply a number and a root by the same number minus the same root, as the root is equal to the number, the product will yield nothing. | ||
| + | |style="text-align:right;"|והסבה למה היא זאת כי ברצותנו לכפול מספר ושרש במספר כמהו פחות שרש אחר כמהו בהיות השרש {{#annot:term|429,1247|2p0q}}שוה{{#annotend:2p0q}} ‫<ref>11v</ref>אל המספר לא יעלה דבר הכפל | ||
| + | |- | ||
| + | | | ||
| + | :For example: suppose you wish to multiply 2 plus a root of 4 by 2 minus a root of 4. | ||
| + | :<math>\scriptstyle\left(2+\sqrt{4}\right)\times\left(2-\sqrt{4}\right)</math> | ||
| + | |style="text-align:right;"|והנה המשל נניח שרצית לכפול ב' ושרש ד' בב' פחות שרש ד‫' | ||
| + | |- | ||
| + | | | ||
| + | :It yields nothing, a nulla [zero] in foreign language | ||
| + | |style="text-align:right;"|ועושה מאומה נוּלַא בלעז | ||
| + | |- | ||
| + | | | ||
| + | :Since a root of 4 is the same as 2. | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{4}=2}}</math> | ||
| + | |style="text-align:right;"|מפני כי ככה הוא שרש ד' כמו שהוא ב‫' | ||
| + | |- | ||
| + | | | ||
| + | :Hence, saying a root of 4 minus [2] is the same as saying nothing. | ||
| + | :<math>\scriptstyle{\color{blue}{2-\sqrt{4}=0}}</math> | ||
| + | |style="text-align:right;"|ולכן באמור שרש ד' פחות שוה לאומר מאומה | ||
| + | |- | ||
| + | | | ||
| + | :Therefore, it is impossible to multiply 2 plus a root of 4 by 2 minus a root of 4. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(2+\sqrt{4}\right)\times\left(2-\sqrt{4}\right)=\varnothing}}</math> | ||
| + | |style="text-align:right;"|ולכן אי אפשר לכפול ב' ושרש ד' בב' פחות שרש ד‫' | ||
| + | |- | ||
| + | | | ||
| + | :If the root is also 2, it is as saying 2 plus 2 by 2 minus 2, which is 2 plus 2 [by] nothing. | ||
| + | |style="text-align:right;"|וזה השרש אם ג"כ ב' שהוא כאומר ב' וב' בב' פחות ב' והינו ב' וב' פחות מאומה | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{\left(2+\sqrt{4}\right)\times\left(2-\sqrt{4}\right)=\left(2+2\right)\times\left(2-2\right)=\left(2+2\right)\times0}}</math> | ||
| + | |- | ||
| + | |The same results from two equal roots. | ||
| + | |style="text-align:right;"|וכדומה לזה יגיע מב' שרשי' שוים | ||
| + | |- | ||
| + | |Therefore, when you wish to divide by such divisors, one should sum the number with the root that is equal to it in one sum and to divide by that sum. | ||
| + | |style="text-align:right;"|ולכן ברצותך לחלק ב{{#annot:term|604,1221|eN1F}}חלוקות{{#annotend:eN1F}} כאלה צריך לחבר המספר עם השרש {{#annot:term|461,1247|3Nbo}}השוה לו{{#annotend:3Nbo}} בסך אחד ולחלק בסך ההוא | ||
| + | |- | ||
| + | |Also to sum the roots that are equal together in one sum and divide by that sum. | ||
| + | |style="text-align:right;"|וכן צריך לחבר השרשי' הבלתי שוים יחד בסך אחד ולחלק בסך ההוא | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== <span style=color:Green>Division of a Number and a Root by a Number and a Root</span> ==== | ||
| + | | | ||
| + | |- | ||
| + | |If you wish to divide a number and a root by a number and a root. | ||
| + | |style="width:45%;text-align:right;"|<big>ואם</big> רצית לחלק מספר ושרש במספר ושרש | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:(19+√25)÷(5+√9)|740|B0XU}}Suppose you wish to divide 19 and a root of 25 by 5 and a root of 9. | ||
| + | :<math>\scriptstyle\left(19+\sqrt{25}\right)\div\left(5+\sqrt{9}\right)</math> | ||
| + | |style="text-align:right;"|<s>ואז</s> ונניח שרצית לחלק י"ט ושרש כ"ה בה' ושרש ט‫'{{#annotend:B0XU}} | ||
| + | |- | ||
| + | | | ||
| + | :Remember that you should multiply 5 plus a root of 9 by 5 minus a root of 9; the result is 16. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(5+\sqrt{9}\right)\sdot\left(5-\sqrt{9}\right)=16}}</math> | ||
| + | |style="text-align:right;"|תזכור שתהיה רוצה לכפול ה' ושרש ט' בה' פחות שרש ט' ועולה י"ו | ||
| + | |- | ||
| + | | | ||
| + | :Say: if we get 5 minus a root of 9 from 16, how much results from 19 and a root of 25. | ||
| + | :<math>\scriptstyle{\color{blue}{16:\left(5-\sqrt{9}\right)=\left(19+\sqrt{25}\right):a}}</math> | ||
| + | |style="text-align:right;"|ואמור אם מי"ו יגיע לנו ה' פחות שרש ט' כמה יגיע מי"ט ושרש <s>י"ט</s> מכ"ה | ||
| + | |- | ||
| + | | | ||
| + | :Multiply 5 plus a root of 9 by 19 plus a root of 25; the result is 95 plus a root of 625, minus a root of 3249, minus a root of 225. | ||
| + | |style="text-align:right;"|תכפול ה' ושרש ט' בי"ט ושרש כ"ה ועולה צ"ה ושרש תרכ"ה פחות שרש ג' אלפי' רמ"ט ופחות שרש רכ"ה | ||
| + | |- | ||
| + | | | ||
| + | :Divide it by 16; the result is 5 and 5 parts of 16 plus a root of 2 and 113 parts of 256, minus a root of 12 and 177 parts of 256. | ||
| + | |style="text-align:right;"|ואלו תחלק בי"ו ויעלה ה' וט"ו חלקי' מי"ו ושרש ב' וקי"ג חלקי' מרנ"ו פחות שרש י"ב וקע"ז חלקי' מרנ"ו | ||
| + | |- | ||
| + | | | ||
| + | :We convert this number into integers; they are 3 and this is the result of division of 19 and a root of 25 by 5 and a root of 9. | ||
| + | |style="text-align:right;"|וזה החשבון {{#annot:term|1071,1431|QYBE}}השיבונו ל{{#annotend:QYBE}}שלמים והיו ג‫'<br> | ||
| + | וככה {{#annot:term|783,1240|rNkJ}}עולה בחלוק{{#annotend:rNkJ}} י"ט ושרש כ"ה בה' ושרש ט‫' | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle\left(19+\sqrt{25}\right)\div\left(5+\sqrt{9}\right)&\scriptstyle=\frac{\left(5{\color{red}{-}}\sqrt{9}\right)\sdot\left(19+\sqrt{25}\right)}{16}=\frac{\left(95+\sqrt{625}\right)-\left(\sqrt{3249}+\sqrt{225}\right)}{16}\\&\scriptstyle=\left(5+\frac{15}{16}\right)+\sqrt{2+\frac{113}{256}}-\sqrt{12+\frac{177}{256}}{\color{red}{-\sqrt{\frac{225}{256}}}}=3\\\end{align}}}</math> | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== <span style=color:Green>Division of a Number by Three Roots</span> ==== | ||
| + | | | ||
| + | |- | ||
| + | |If you wish to divide a certain number by three roots: | ||
| + | |style="width:45%;text-align:right;"|<big>ואם</big> רצית לחלק בג' שרשי' מספר מה | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:36÷(√4+√9+√16)|740|UR1i}}Suppose you wish to divide 36 by a root of 4, a root of 9, and a root of 16. | ||
| + | :<math>\scriptstyle36\div\left(\sqrt{4}+\sqrt{9}+\sqrt{16}\right)</math> | ||
| + | |style="text-align:right;"|ונניח שרצית לחלק ל"ו בשרש ד' ובשרש ט' ובשרש י"ו בדבר{{#annotend:UR1i}} | ||
| + | |- | ||
| + | | | ||
| + | :Say as if these roots are [in]expressible: you should multiply a root of 4, a root of 9, and a root of 16, by a root of 4 plus a root of 9 minus a root of 16; the result is a root of 144 minus 3. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(\sqrt{4}+\sqrt{9}+\sqrt{16}\right)\sdot\left(\sqrt{4}+\sqrt{9}-\sqrt{16}\right)=\sqrt{144}-3}}</math> | ||
| + | |style="text-align:right;"|ואמור מאלו השרשי' כאלו הם מדוברים אתה צריך לכפול שרש ד' <s>ב</s><sup>ו</sup>שרש ט' ושרש י"ו בשרש ד' ושרש ט' פחות שרש י"ו<br> | ||
| + | שעולה שרש קמ"ד פחות ג' מספרי‫' | ||
| + | |- | ||
| + | | | ||
| + | :Therefore, when dividing a root of 144 minus 3 by a root of 4, a root of 9 and a root of 16, you get a root of 4 plus a root of 9 minus a root of 16, as you saw above in the rule of dividing a number by a root and a number. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(\sqrt{144}-3\right)\div\left(\sqrt{4}+\sqrt{9}+\sqrt{16}\right)=\sqrt{4}+\sqrt{9}-\sqrt{16}}}</math> | ||
| + | |style="text-align:right;"|א"כ לחלוק שרש קמ"ד פחות ג' בשרש ד' ושרש ט' ובשרש י"ו {{#annot:term|875,1575|eaEl}}יגיע לך{{#annotend:eaEl}} שרש ד' ושרש ט' פחות שרש י"ו כפי מה שהראית לפנים בכלל חלוק מספר בשרש ומספר | ||
| + | |- | ||
| + | | | ||
| + | :So, we say that this root minus a number, meaning a root of 144 minus 3, which is the aforementioned product, is the divisor from here on. | ||
| + | :<math>\scriptstyle{\color{red}{\left(\sqrt{4}+\sqrt{9}+\sqrt{16}\right)\sdot\left(\sqrt{4}+\sqrt{9}-\sqrt{16}\right)}}</math> = <math>\scriptstyle{\color{blue}{\sqrt{144}-3}}</math> | ||
| + | |style="text-align:right;"|ולכן נאמר כי זה השרש פחות מספר רצוני שרש מקמ"ד פחות ג' אשר הוא כפל ממה שנאמ' קודם הנה הוא יהיה מכאן ולהבא {{#annot:term|604,1228|lfgo}}מחלק{{#annotend:lfgo}} | ||
| + | |- | ||
| + | | | ||
| + | :We say, according to the rule of three: if a root of 144 minus 3 yields a root of 4 plus a root of 9 minus a root of 16, how much yields 36, which we intended above to divide by the three roots? | ||
| + | :<math>\scriptstyle{\color{blue}{\left(\sqrt{144}-3\right):\left(\sqrt{4}+\sqrt{9}-\sqrt{16}\right)=36:a}}</math> | ||
| + | |style="text-align:right;"|ונאמר בכלל הג' אם שרש קמ"ד פחות ג' נותן שרש ד' ושרש ט' פחות שרש י"ו מה יתן ל"ו אשר אמרנו למעלה לחלוק בג' השרשי‫' | ||
| + | |- | ||
| + | | | ||
| + | :Multiply a root of 4 plus a root of 9 minus a root of 16 by 36; the result is a root of 5184 plus a root of 116[6]4 minus a root of 20736. | ||
| + | |style="text-align:right;"|תכפול שרש ד' ושרש ט' פחות שרש י"ו בל"ו<br> | ||
| + | שעולה שרש מה' אלפי' וקפ"ד ושרש מי"א אלפי' תרי"ד פחות שרש כ' אלפי' ותשל"ו | ||
| + | |- | ||
| + | | | ||
| + | :Divide it by a root of 144 minus 3, which is the divisor. | ||
| + | |style="text-align:right;"|וזה תחלק בשרש קמ"ד פחות ג' אשר זה המחלק | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{36\div\left(\sqrt{4}+\sqrt{9}+\sqrt{16}\right)=\frac{\left(\sqrt{4}+\sqrt{9}-\sqrt{16}\right)\sdot36}{\sqrt{144}-3}=\frac{\sqrt{5184}+\sqrt{116{\color{red}{6}}4}-\sqrt{20736}}{\sqrt{144}-3}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :We can convert it to an expressible number, because 144 has an expressible root: | ||
| + | |style="text-align:right;"|נוכל להשיבו אל מספר מדבר מפני כי קמ"ד יש לו שרש מדבר | ||
| + | |- | ||
| + | | | ||
| + | :So, we say according to the previous method of division by a root minus a number: multiply a root of 144 minus 3 by a root of 144 plus 3; the result is 135. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(\sqrt{144}-3\right)\sdot\left(\sqrt{144}+3\right)=135}}</math> | ||
| + | |style="text-align:right;"|ולכן נאמ' עוד באופן חלוקת הקודם בשרש פחות מספר תכפול שרש מקמ"ד פחות ג' בשרש קמ"ד וג' יותר עולה קל"ה | ||
| + | |- | ||
| + | | | ||
| + | :We say that this product is the divisor. | ||
| + | |style="text-align:right;"|ונאמר מראש שזה הכפל הוא המחלק | ||
| + | |- | ||
| + | | | ||
| + | :We return to the rule of three and say: if [a root of] 144 plus 3 results from 135, how much results from a root of 5184 plus a root of 11664 minus a root of 20736. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{135:\left(\sqrt{144}+3\right)=\left(\sqrt{5184}+\sqrt{11664}-\sqrt{20736}\right):a}}</math> | ||
| + | |style="text-align:right;"|ונשוב אל הכלל מהג' ונאמר אם מקל"ה יגיע קמ"ד וג' יותר כמה {{#annot:term|875,1575|aPlU}}יגיע מ{{#annotend:aPlU}}שרש ה' אלפי' וקפ"ד ומשרש י"א אלפי' תרס"ד פחות שרש כ' אלפי' תשל"ו | ||
| + | |- | ||
| + | | | ||
| + | :Multiply a root of 144 plus 3 by a root of 5184, plus a root of 11664, minus a root of 20736; the result is a root of 746496, plus a root of 1679616, a root of 46656, and a root of 104976, minus a root of 2985984, minus a root of 186624. | ||
| + | |style="text-align:right;"|‫<ref>12r</ref>תכפול שרש קמ"ד וג' יותר בשרש ה' אלפי' וקפ"ד ושרש מי"א אלפי' תרס"ד פחות שרש מכ' אלפי' תשל"ו<br> | ||
| + | ועולה שרש מתשמ"ו אלפי' ותצ"ו ושרש מאלף ותרע"ט אלפי' ותרי"ו ושרש מ"ו אלפי' ותרנ"ו ושרש ק"ד אלפי' ותתקע"ו פחות שרש מ 4895892 ופחות שרש מ 426681 | ||
| + | |- | ||
| + | | | ||
| + | :Divide this product by 135, returned to a root; the result is a root of 40 and 24 parts of 25, a root of 92 and 4 parts of 25, a root of 2 and 14 parts of 25, a root of 5 and 19 parts of 25, minus a root of 163 and 21 parts of 25, minus a root of ten and 6 parts of 25. | ||
| + | |style="text-align:right;"|וזה הכפל תחלק בקל"ה {{#annot:term|1071,2559|Ycae}}מושב ל{{#annotend:Ycae}}שרש שעולה שרש מ' וכ"ד חלקי' מכ"ה ושרש צ"ב וד' חלקי' מכ"ה ושרש ב' וי"ד חלקי' מכ"ה ושרש ה' וי"ט חלקי' מכ"ה פחות שרש קס"ג וכ"א חלקי' מכ"ה ופחות שרש עשרה וו' חלקי' מכ"ה | ||
| + | |- | ||
| + | | | ||
| + | :This is the result of division of 36 by a root of 4, a root of 9 and a root of 16 and when these roots are converted to expressible numbers the total is 4. | ||
| + | |style="text-align:right;"|וככה עולה <s>וכ"א חלקי' מכ"ה</s> לחלוק ל"ו בשרש ד' ובשרש ט' ובשרש י"ו אשר אלו השרשי' כאשר {{#annot:term|1071,2559|HBuz}}הושבו אל{{#annotend:HBuz}} {{#annot:term|1075,2192|orLt}}מספרי' מדוברי'{{#annotend:orLt}} יהיו בסך ד‫' | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle36\div\left(\sqrt{4}+\sqrt{9}+\sqrt{16}\right)&\scriptstyle=\frac{\left(\sqrt{144}+3\right)\sdot\left(\sqrt{5184}+\sqrt{11664}-\sqrt{20736}\right)}{135}\\&\scriptstyle=\frac{\sqrt{746496}+\sqrt{1679616}+\sqrt{46656}+\sqrt{104976}-\sqrt{2985984}-\sqrt{186624}}{135}\\&\scriptstyle=\sqrt{40+\frac{24}{25}}+\sqrt{92+\frac{4}{25}}+\sqrt{2+\frac{14}{25}}+\sqrt{5+\frac{19}{25}}-\sqrt{163+\frac{21}{25}}-\sqrt{10+\frac{6}{25}}\\\end{align}}}</math> | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== <span style=color:Green>Division of a Number by Four Roots</span> ==== | ||
| + | | | ||
| + | |- | ||
| + | |If you wish to divide a certain number by four roots: | ||
| + | |style="width:45%;text-align:right;"|<big>ואם</big> רצית לחלק מספר מה בד' שרשי‫' | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:70÷(√4+√9+√16+√25)|740|cUYO}}Suppose you wish to divide 70 by the sum of a root of 4, a root of 9, a root of 16, and a root of 25, as if these roots were inexpressible. | ||
| + | :<math>\scriptstyle70\div\left(\sqrt{4}+\sqrt{9}+\sqrt{16}+\sqrt{25}\right)</math> | ||
| + | |style="text-align:right;"|ונניח שרצית לחלק ע' בשרש ד' ובשרש ט' ובשרש י"ו ובשרש כ"ה מחוברי' כלם יחד באופן כאלו היו השרשי' האלו בלתי מדוברי‫'{{#annotend:cUYO}} | ||
| + | |- | ||
| + | |You should create one product from these four roots, always placing the greater root first, as you have seen, this way: | ||
| + | |style="text-align:right;"|הנך צריך {{#annot:term|185,1426|yJC5}}לעשות כפל{{#annotend:yJC5}} אחד מאלו הד' שרשי' בשומך לעולם הגדול מהשרשי' קודם כפי מה שהראית באופן זה | ||
| + | |- | ||
| + | | | ||
| + | :As saying: a root of 25, a root of 16, a root of 9, and a root of 4, by a root of 25 plus a root of 16 minus a root of 9, and minus a root of 4. | ||
| + | :The result is [28] plus a root of 1600 minus a root of 144. | ||
| + | |style="text-align:right;"|כאמור שרש כ"ה ושרש י"ו ושרש ט' ושרש ד' בשרש כ"ה ושרש י"ו פחות שרש ט' ופחות שרש ד‫'<br> | ||
| + | ועולה שרש כ"ח ושרש אלף ת"ר פחות שרש קמ"ד | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{\left(\sqrt{25}+\sqrt{16}+\sqrt{9}+\sqrt{4}\right)\sdot\left(\sqrt{25}+\sqrt{16}-\sqrt{9}-\sqrt{4}\right)={\color{red}{28}}+\sqrt{1600}-\sqrt{144}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :We say that when dividing 28 plus a root of 1600 minus a root of 144 by a root of 25, a root of 16, a root of 9, and a root of 4, the result is a root of 25 plus a root of 16 minus a root of 9, and minus a root of 4. | ||
| + | :<math>\scriptstyle{\color{blue}{\frac{28+\sqrt{1600}-\sqrt{144}}{\sqrt{25}+\sqrt{16}+\sqrt{9}+\sqrt{4}}=\sqrt{25}+\sqrt{16}-\sqrt{9}-\sqrt{4}}}</math> | ||
| + | |style="text-align:right;"|ונאמר כי בחלוק כ"ח ושרש אלף ת"ר פחות שרש קמ"ד בשרש כ"ה ובשרש י"ו ובשרש ט' ובשרש ד' יעלה שרש כ"ה ושרש י"ו פחות שרש ט' ופחות שרש ד‫' | ||
| + | |- | ||
| + | | | ||
| + | :I ask, then: how much is obtained from 70? | ||
| + | |style="text-align:right;"|אשאל א"כ כמה יעלה מע‫' | ||
| + | |- | ||
| + | | | ||
| + | :You should multiply according to the rule of three: 70 by a root of 25 plus a root of 16 minus a root of 9, and minus a root of 4. | ||
| + | :The result is a root of 122500 plus a root of 78400 minus a root of 44100 and minus a root of 19600. | ||
| + | |style="text-align:right;"|הנך צריך לכפול כפי הכלל מהג' ע' בשרש כ"ה ושרש י"ו פחות שרש ט' ופחות שרש ד‫'<br> | ||
| + | שעולה שרש מקכ"ב אלפי' ות"ק ושרש מע"ח אלפי' ות' פחות שרש מ"ד אלפי' וק' ופחות שרש מי"ט אלפי' ות"ר | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{70\sdot\left(\sqrt{25}+\sqrt{16}-\sqrt{9}-\sqrt{4}\right)=\sqrt{122500}+\sqrt{78400}-\sqrt{44100}-\sqrt{19600}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :Then, you should divide this product by 28 plus a root of 1600 minus a root of 144. | ||
| + | |style="text-align:right;"|וזה הכפל הנך צריך לחלק בכ"ח ושרש אלף ות"ר פחות שרש קמ"ד | ||
| + | |- | ||
| + | | | ||
| + | :This [divisor] consists of three terms, "klapi" in a foreign language, so you should proceed according to the rule of the division of three roots and multiply 28 plus a root of 1600 minus a root of 144 by 28 and a root of 1600 plus a root of 144. | ||
| + | :The result is 2240 and a root of 5017600. | ||
| + | |style="text-align:right;"|וזה החלוק הוא ג' [{{#annot:term|1578,1404|bBb3}}קשרים{{#annotend:bBb3}}]‫<ref>marg.</ref> {{#annot:term|1578|g8EG}}קלאפי{{#annotend:g8EG}} בלעז ולכן תצטרך להמשך כפי הכלל מהחלוק בג' שרשים ו{{#annot:term|185,1426|KZ2l}}לעשות{{#annotend:KZ2l}} שרש כ"ח ושרש אלף ת"ר פחות שרש קמ"ד בכ"ח ושרש אלף ת"ר ויותר שרש מקמ"ד<br> | ||
| + | שעולה אלפיים ור"מ ושרש מ 0067105 | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{\left(28+\sqrt{1600}-\sqrt{144}\right)\sdot\left(28+\sqrt{1600}+\sqrt{144}\right)=2240+\sqrt{5017600}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :When this product is divided by 28 plus a root of 1600 minus a root of 144; the result is 28 and a root of 1600 plus a root of 144. | ||
| + | :<math>\scriptstyle{\color{blue}{\frac{2240+\sqrt{5017600}}{28+\sqrt{1600}-\sqrt{144}}=28+\sqrt{1600}+\sqrt{144}}}</math> | ||
| + | |style="text-align:right;"|וזה הכפל כאשר {{#annot:term|784,1226|Orfk}}נחלק ב{{#annotend:Orfk}}כ"ח ושרש אלף ת"ר פחות שרש קמ"ד יעלה כ"ח ושרש אלף ת"ר ויותר שרש קמ"ד | ||
| + | |- | ||
| + | | | ||
| + | :I ask: how much is the result of a root of 122500 plus a root of 78400 minus a root of 44100 and minus a root of 19600? | ||
| + | |style="text-align:right;"|אשאל כמה יעלה משרש קכ"ב אלפי' ות"ק ושרש ע"ח אלפי' ות' פחות שרש מ"ד אלפי' וק' ופחות שרש י"ט אלפי' ות"ר | ||
| + | |- | ||
| + | | | ||
| + | :You should multiply according to the rule of three: 28 and a root of 1600 plus a root of 144 by a root of 122500 plus a root of 78400 minus a root of 44100 and minus a root of 19600. | ||
| + | :The result is a root of 96040000, a root of 61465600, a root of 196000000, a root of 125440000, a root of 17640000, and a root of 11289600, minus a root of 34574400, minus a root of 15366400, minus a root of 70560000, minus a root of 31360000, minus a root of 6350400, and minus a root of 2822400. | ||
| + | |style="text-align:right;"|הנך צריך לכפול כפי הכלל מהג' כ"ח ושרש אלף ת"ר ועוד שרש קמ"ד בשרש קכ"ב אלפי' ות"ק ושרש ע"ח אלפי' ות' פחות שרש ממ"ד אלפי' וק' ופחות שרש מי"ט אלפי' ת"ר<br> | ||
| + | שעולה שרש מ 00004069 ושרש <s>00656416</s> מ 00656416 ושרש מ 000000691 ושרש מ 000044521 ושרש מ 00004671 ושרש מ 00698211 פחות שרש מ 00447543 ופחות שרש מ 00466351 ופחות שרש מ 00006507 ופחות שרש מ 00006313 ופחות שרש מ 0040536 ופחות שרש מ 0042282 | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{\left(28+\sqrt{1600}+\sqrt{144}\right)\sdot\left(\sqrt{122500}+\sqrt{78400}-\sqrt{44100}-\sqrt{19600}\right)=}}</math> | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{96040000}+\sqrt{61465600}+\sqrt{196000000}+\sqrt{125440000}+\sqrt{17640000}+\sqrt{11289600}}}</math> | ||
| + | :<math>\scriptstyle{\color{blue}{-\sqrt{34574400}-\sqrt{15366400}-\sqrt{70560000}-\sqrt{31360000}-\sqrt{6350400}-\sqrt{2822400}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :You divide this product by 2240 plus a root of 5017600 - this divisor consists of two terms, "klapi" in a foreign language, that are equal. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(2240+\sqrt{5017600}\right)\longrightarrow2240=\sqrt{5017600}}}</math> | ||
| + | |style="text-align:right;"|וזה הכפל תחלק באלפיים ר"מ ושרש מ 0067105 אשר זה המחלק הוא ‫<ref>12v</ref>מב' קשרי' קלאפי בלעז שהם שוים | ||
| + | |- | ||
| + | | | ||
| + | :If one of them was greater than the other, we would have applied the method of division by two roots. | ||
| + | |style="text-align:right;"|ואם היה אחד מהם גדול מהאחר היינו מתעסקי' בזה באופן <sup>ה</sup>חלוק <s>ב</s><sup>בב'</sup> שרשי‫' | ||
| + | |- | ||
| + | | | ||
| + | :But, it is impossible to continue according to what is said previously, because it is necessary to combine the terms into one expression, since they [are] equal. | ||
| + | |style="text-align:right;"|אבל אי אפשר עתה להמשך כפי מה שנאמר קודם מפני כי צריך לחבר החלקי' יחד בקול אחד כאשר הם בלתי שוים | ||
| + | |- | ||
| + | | | ||
| + | :So, add 2240 to a root of 5017600, which is 2240. | ||
| + | :The result is 4480 and this is the said divisor. | ||
| + | :<math>\scriptstyle{\color{blue}{2240+\sqrt{5017600}=4480}}</math> | ||
| + | |style="text-align:right;"|לכן תחבר אלפיים ור"מ עם שרש מ 0067105 שהוא אלפיים ור"מ<br> | ||
| + | ועולה ד' אלפי' ות"פ וככה הוא המחלק האמור | ||
| + | |- | ||
| + | | | ||
| + | :Divide, then, the product mentioned above by twelve terms, which are six roots minus six other roots; the result is a root of 4 and 201 parts of 256, a root of 3 and 16 parts of 256, a root of 9 and 196 parts of 256, a root of 6 and 64 parts of 256, a root of 0 and 225 parts of 256, and a root of 0 and 144 parts of 256, minus a root of 1 and 185 parts of 256, minus a root of 0 and 196 parts of 256, minus a root of 3 and 132 parts of 256, minus a root of 1 and 144 parts of 256, minus a root of 0 and 81 parts of 256, minus a root of 0 and 36 parts of 256; and this is the result of division of 70 by a root of 4, a root of 9, a root of 16, and a root of 25. | ||
| + | |style="text-align:right;"|אם כן תחלק הכפל האמור <s>לפנים</s> קודם בי"ב {{#annot:term|1578,1404|EM5u}}קשרים{{#annotend:EM5u}} שהם ו' שרשי' פחות ו' שרשי' אחרים שיעלה שרש ד' ור"א חלקי' מרנ"ו ושרש ג' וי"ו חלקי' מרנ"ו ושרש ט' וקצ"ו חלקי' מרנ"ו ושרש ו' וס"ד חלקי' מרנ"ו ושרש 0 ורכ"ה חלקי' מרנ"ו ושרש 0 וקמ"ד חלקי' מרנ"ו פחות שרש א' וקפ"ה חלקי' מרנ"ו ופחות שרש 0 וקצ"ו חלקי' מרנ"ו ופחות שרש ג' וקל"ב חלקי' מרנ"ו ופחות שרש א' וקמ"ד חלקי' מרנ"ו ופחות שרש 0 ופ"א חלקי' מרנ"ו ופחות שרש 0 ול"ו חלקי' מרנ"ו<br> | ||
| + | וככה {{#annot:term|783,1240|avzC}}יעלה לחלוק{{#annotend:avzC}} ע' בשרש ד' ובשרש ט' ובשרש י"ו ובשרש כ"ה | ||
| + | |- | ||
| + | | | ||
| + | :When these division roots are converted into an expressible root, their sum is 5 integers. | ||
| + | |style="text-align:right;"|ואלו שרשי החלוקה כאשר הושבו ל{{#annot:term|1075,2192|npuB}}מספר מדובר{{#annotend:npuB}} יהיה סכומם ה' מספרים שלמים | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle70\div &\scriptstyle\left(\sqrt{4}+\sqrt{9}+\sqrt{16}+\sqrt{25}\right)=\\&\scriptstyle=\sqrt{4+\frac{201}{256}}+\sqrt{3+\frac{16}{256}}+\sqrt{9+\frac{196}{256}}+\sqrt{6+\frac{64}{256}}+\sqrt{0+\frac{225}{256}}+\sqrt{0+\frac{144}{256}} | ||
| + | \\&\scriptstyle-\sqrt{1+\frac{185}{256}}-\sqrt{0+\frac{196}{256}}-\sqrt{3+\frac{132}{256}}-\sqrt{1+\frac{144}{256}}-\sqrt{0+\frac{81}{256}}-\sqrt{0+\frac{36}{256}}\\&\scriptstyle=5\\\end{align}}}</math> | ||
| + | |- | ||
| + | |It is also possible to divide it by the stated roots or by any other four roots in a different way that seems more difficult at first than the way you saw, but it is easier later in the procedure, and it is required when you wish to divide by more roots. This is the way: | ||
| + | |style="text-align:right;"|<big>עוד</big> אפשר לחלקו בשרשים האמורי' או בד' שרשי' אחרים שיזדמנו באופן אחר הנראה יותר חמור בהתחלה <sup>מן</sup> האופן אשר הראית אבל הוא יותר נקל בהמשך {{#annot:term|469,1656|qnF0}}הפעל{{#annotend:qnF0}} ג"כ הוא מוצרך ברצותך לחלק ביותר שרשים וזה הוא זה האופן | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:70÷(√4+√9+√16+√25)|740|W9ga}}Suppose you wish to divide 70 by the sum of a root of 4, a root of 9, a root of 16, and a root of 25, as if the roots were inexpressible. | ||
| + | :<math>\scriptstyle70\div\left(\sqrt{4}+\sqrt{9}+\sqrt{16}+\sqrt{25}\right)</math> | ||
| + | |style="text-align:right;"|נניח שרצית לחלק ע' בשרש ד' ובשרש ט' ובשרש י"ו ובשרש כ"ה מחוברי' באופן כאלו היו שרשי' בלתי מדברי‫'{{#annotend:W9ga}} | ||
| + | |- | ||
| + | |Sum up the two smaller together and the two greater together in a way they can be summed, if any of them cannot be combined into one expression. | ||
| + | |style="text-align:right;"|תחבר שני הקטני' יחד ושני הגדולים יחד באופן שאפשר לחברם אם לא יתכן חבורם איזה מהם יחד בקול אחד | ||
| + | |- | ||
| + | |Assuming that when one is multiplied by the other the result is an inexpressible root: | ||
| + | |style="text-align:right;"|בהניח כי כשיכפל האחד באחר יעשה שרש בלתי מדבר | ||
| + | |- | ||
| + | | | ||
| + | :When summing the root of 4 to the root of 9 by the mentioned method, it yields a root of the sum of a root of 144 plus 13. | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{4}+\sqrt{9}=\sqrt{\sqrt{144}+13}}}</math> | ||
| + | |style="text-align:right;"|ולכן בחבר שרש ד' בשרש ט' באופן האמור עושה שרש מחבור שרש קמ"ד עם י"ג | ||
| + | |- | ||
| + | | | ||
| + | :Then, you sum up the root of 16 with the root of 25; the result is a root of the sum of a root of 1600 plus 41, when it is added to it in the aforementioned way, i.e. in the second way for those that cannot be expressed in one expression. | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{16}+\sqrt{25}=\sqrt{\sqrt{1600}+41}}}</math> | ||
| + | |style="text-align:right;"|אח"כ תחבר שרש י"ו עם שרש כ"ה ועושה שרש מחבור שרש אלף ת"ר עם מ"א {{#annot:term|178,1165|nw9m}}בחבר אליו{{#annotend:nw9m}} באופן האמור קודם דהיינו באופן השני מאלו אשר אי אפשר לחברם בקול אחד | ||
| + | |- | ||
| + | |Place the two greater roots before the two smaller as they are summed. | ||
| + | |style="text-align:right;"|אח"כ תשים שני השרשי' הגדולי' קודם שני הקטני' כפי מה שהם מחוברי‫' | ||
| + | |- | ||
| + | |Multiply them by the two greater addends minus the two smaller addends. | ||
| + | |style="text-align:right;"|ותכפלם בשני הגדולי' מחוברי' פחות שני הקטני' מחוברי' באלו | ||
| + | |- | ||
| + | | | ||
| + | :Saying: a root of a sum of [a root of] 1600 with 41, plus a root of a sum of a root of 144 with 13, by a root of a sum of a root of 1600 with 41, minus a root of a sum of [a root of] 144 with 13. | ||
| + | :The result is 28 plus a root of 1600 minus a root of 144. | ||
| + | |style="text-align:right;"|באמור שרש מחבור אלף ת"ר עם מ"א ושרש מחבור שרש קמ"ד עם י"ג בשרש מחבור שרש אלף ת"ר עם <s>י"ג</s> מ"א פחות שרש מחבור קמ"ד עם י"ג<br> | ||
| + | ועולה כ"ח ושרש אלף ת"ר פחות שרש קמ"ד | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{\left(\sqrt{\sqrt{1600}+41}+\sqrt{\sqrt{144}+13}\right)\sdot\left(\sqrt{\sqrt{1600}+41}-\sqrt{\sqrt{144}+13}\right)=28+\sqrt{1600}-\sqrt{144}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :Now, return to the rule of three and say: if this product, i.e. 28 plus a root of 1600 minus a root of 144, yields a root of the sum of a root of 1600 plus 41 minus a root of the sum of [a root of] 144 plus 13, how much does 70 yield? | ||
| + | |style="text-align:right;"|עתה תשוב אל הכלל מהג' ואמור אם מזה הכפל רצוני מכ"ח ושרש אלף ת"ר פחות שרש קמ"ד יגיע שרש מחבור שרש מאלף ת"ר עם מ"א פחות שרש מחבור קמ"ד עם י"ג כמה יעלה מע‫' | ||
| + | |- | ||
| + | | | ||
| + | :Multiply a root of a sum of [a root of] 1600 with 41 minus a root of a sum of a root of 144 with 13 by 70. | ||
| + | :The result is a root of a sum of a root of 38416000000 with 200900 minus a root of a sum of a root of 3457440000 with 63700. | ||
| + | |style="text-align:right;"|תכפול שרש מחבור אלף ת"ר עם מ"א פחות שרש מחבור שרש קמ"ד עם י"ג בע‫'<br> | ||
| + | שעולה שרש מחבור שרש מ 00000061483 עם שרש מ 009002 פחות שרש מחבור שרש מ 0000447543 עם 00736 | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{70\sdot\left(\sqrt{\sqrt{1600}+41}-\sqrt{\sqrt{144}+13}\right)=\sqrt{\sqrt{38416000000}+200900}-\sqrt{\sqrt{3457440000}+63700}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :Divide it by 28 plus a root of 1600 minus a root of 144; this divisor consists of three terms. | ||
| + | :<math>\scriptstyle{\color{blue}{\div\left(28+\sqrt{1600}-\sqrt{144}\right)}}</math> | ||
| + | |style="text-align:right;"|וזה תחלק ‫<ref>13r</ref>על כ"ח ושרש אלף ת"ר פחות שרש קמ"ד אשר זה המחלק הוא מג' קשרים | ||
| + | |- | ||
| + | |It should be continued according to the rule of division by three as we showed above. | ||
| + | |style="text-align:right;"|וצריך להמשך כפי כלל החלוק בג' כפי מה שהראינו קודם | ||
| + | |- | ||
| + | |You can also divide by these three terms according to the way we introduced above in this chapter. | ||
| + | |style="text-align:right;"|וג"כ תוכל לחלק באלו ג' קשרים כפי האופן אשר המשכנו למעלה בזה הכלל | ||
| + | |- | ||
| + | | | ||
| + | :Meaning, by summing 28, which is a root of 784, with a root of 1600. | ||
| + | :The result is a root of a sum of [a root of] 5017600 with 2384. | ||
| + | :<math>\scriptstyle{\color{blue}{28+\sqrt{1600}=\sqrt{784}+\sqrt{1600}=\sqrt{\sqrt{5017600}+2384}}}</math> | ||
| + | |style="text-align:right;"|רצוני בחבר כ"ח שהוא שרש מתשפ"ד עם שרש אלף ת"ר<br> | ||
| + | שעולה שרש מחבור מ 0067105 עם 4832 | ||
| + | |- | ||
| + | | | ||
| + | :This root is summed with a subtractive root, which is a root of 144. | ||
| + | :<math>\scriptstyle{\color{blue}{28+\sqrt{1600}-\sqrt{144}=\sqrt{\sqrt{5017600}+2384}-\sqrt{144}}}</math> | ||
| + | |style="text-align:right;"|אשר זה השרש א' סכום יש לו שרש אחד פחות דהיינו שרש קמ"ד | ||
| + | |- | ||
| + | | | ||
| + | :So, multiply a root of a sum of a root of 5017600 with 2384 minus a root of 144 by a root of a sum of a root of 5017600 with 2384 plus a root of 144. | ||
| + | :The result is 2240 plus a root of 5017600. | ||
| + | |style="text-align:right;"|ולכן תכפול שרש מחבור שרש מ 00671<sup>0</sup>5 עם שרש מ 4832 פחות שרש קמ"ד בשרש מחבור שרש מ 0067105 עם 4832 ויותר שרש מקמ"ד<br> | ||
| + | שעולה 0422 ויותר שרש מ 0067105 | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{\left(\sqrt{\sqrt{5017600}+2384}-\sqrt{144}\right)\sdot\left(\sqrt{\sqrt{5017600}+2384}+\sqrt{144}\right)=2240+\sqrt{5017600}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :Now, you should return to the rule of three again and say: if 2240 plus a root of 5017600 yields a root of a sum of a root of 5017600 with 2[3]84 plus a root of 144, how much does a root of a sum of [a root of] 38416000000 with 200900 minus a root of a sum of a root of 3457440000 with 63700 yield? | ||
| + | |style="text-align:right;"|עתה צריך אתה <s>להשיב</s> <sup>לשוב</sup> עוד לכלל הג' ואמור אם מאלפיים ור"מ ויותר שרש מ 0067105 יגיע שרש מחבור שרש מ 0067105 עם אלפיים רפ"ד ויותר שרש קמ"ד כמה יגיע משרש חבור מ 00000061483 עם 009002 פחות שרש מחבור שרש מ 0000447543 עם 00736 | ||
| + | |- | ||
| + | | | ||
| + | :Multiply a root of a sum of a root of 5017600 with 2384, plus a root of 144, by a root of a sum of a root of 38416000000 with 200900, minus a root of a sum of a root of 3457440000 with 63700. | ||
| + | :The result is a root of a sum of a root of 192756121600000000 with a root of 202514400256000000, | ||
| + | :plus a root of a sum of a root of 218335645696000000 with 478945600, | ||
| + | :plus a root of a sum of a root of 796594[1]76000000 [with 28929600, | ||
| + | :minus a root of a sum of a root of [17348050944000000] with 20359865344000000, | ||
| + | :minus a root of a sum of a root of 19650208112640000 with 151860800, | ||
| + | :minus a root of a sum of a root of 71693475840000 with 9172800. | ||
| + | |style="text-align:right;"|תכפול שרש מחבור שרש מ 0067105 עם שרש אלפיים ושפ"ד ויותר שרש קמ"ד בשרש מחבור שרש מ 00000061483 עם 009002 פחות שרש מחבור <s>ש</s> 0000447543 עם 00736<br> | ||
| + | שעולה שרש מחבור שרש מ 000000006121657291 עם שרש מחבור שרש מ 000000652004415202<br> | ||
| + | ועם שרש מחבור שרש מ 000000696546533812 עם 006549874<br> | ||
| + | ויותר שרש מחבור שרש מ 00000067495697<br> | ||
| + | עם שרש מחבור שרש מן 00000044356895302<br> | ||
| + | ועם שרש מחבור שרש מ 00004621180205691 עם 008068151<br> | ||
| + | ופחות שרש מחבור שרש מ 00004857439617 עם 0082719 | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle\left(\sqrt{\sqrt{5017600}+2384}+\sqrt{144}\right)\sdot&\scriptstyle\left(\sqrt{\sqrt{38416000000}+200900}-\sqrt{\sqrt{3457440000}+63700}\right)=\\&\scriptstyle=\sqrt{\sqrt{192756121600000000}+\sqrt{202514400256000000}}\\&\scriptstyle+\sqrt{\sqrt{218335645696000000}+478945600}\\&\scriptstyle+\sqrt{\sqrt{796594{\color{red}{1}}76000000}+{\color{red}{28929600}}}\\&\scriptstyle-\sqrt{{\color{red}{\sqrt{17348050944000000}}}+\sqrt{20359865344000000}}\\&\scriptstyle{\color{red}{-}}\sqrt{\sqrt{19650208112640000}+151860800}\\&\scriptstyle-\sqrt{\sqrt{71693475840000}+9172800}\\\end{align}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :Divide this product by 2240 plus a root of 5017600, as the divisor consists of two terms. | ||
| + | |style="text-align:right;"|וזה הכפל תחלק ב 0422 ויותר שרש מ 0067105 אשר המחלק הוא מב' קשרים | ||
| + | |- | ||
| + | |The procedure should continue according to the rule of division of two roots, if the terms are not equal. | ||
| + | |style="text-align:right;"|וצריך להמשיך הענין כפי הכלל מהחלוק מב' שרשי' אם הקשרי' היו בלתי שוים | ||
| + | |- | ||
| + | | | ||
| + | :But, since they are equal, meaning the root of 5017600 is 2240, we divide by its double, which is 4480. | ||
| + | :<math>\scriptstyle{\color{blue}{\div\left(2240+\sqrt{5017600}\right)=2240+2240=4480}}</math> | ||
| + | |style="text-align:right;"|אבל מפני שהם שוים רצוני ששרש מ 0067105 הוא אלפיים ור"מ אנחנו נחלק ב{{#annot:term|387,1230|vbVH}}כפלו{{#annotend:vbVH}} שהוא ד' אלפים ות"פ | ||
| + | |- | ||
| + | | | ||
| + | :The result is a root of a sum of a root of 478 plus 2112 parts of 4096 with a root of 502 plus 3[03]3 [parts] of 4096. | ||
| + | |style="text-align:right;"|שיגיע ממנו שרש מחבור שרש מתע"ח ואלפיים קי"ב חלקי' מד' אלפי' צ"ו<br> | ||
| + | עם שרש מחבור תק"ב וג' אלפי' וש"ג מד' אלפי' צ"ו | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{\sqrt{478+\frac{2112}{4096}}+\sqrt{502+\frac{3033}{4096}}+{\color{red}{\sqrt{542+\frac{68}{4096}}+23+\frac{221}{256}}}}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :The sum has a root of 91 plus 113 parts of 256; this root is 9 plus 9 parts of 16. | ||
| + | |style="text-align:right;"|ובסכום יהיה שרש מצ"א <s>וי"ג</s> וקי"ג חלקי' מרנ"ו אשר זה השרש הוא ט' וט' חלקי' מי"ו | ||
| + | |- | ||
| + | | | ||
| + | :Plus a root of a sum of a root of 1 and 4004 parts of 4096 with 1 and 113 parts of 256; the result is a root of 2 plus 217 parts of 256; this root is 1 plus 11 parts of 16. | ||
| + | |style="text-align:right;"|ויותר שרש מחבור שרש א' וד' אלפי' וד' חלקי' מד' אלפי' וצ"ו עם א' וקי"ג חלקי' מרנ"ו שעולים לסך שרש ב' ורי"ז חלקי' מרנ"ו אשר זה השרש הוא א' וי"א חלקי' מי"ו | ||
| + | |- | ||
| + | | | ||
| + | :When it is summed with the former root, which is 9 plus 9 parts of 16, the result is 11 and a quarter. | ||
| + | |style="text-align:right;"|ובחברם עם השרש הראשון שהוא ט' וט' חלקי' מי"ו ועולה י"א ורביע | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{91+\frac{113}{256}}+\sqrt{\sqrt{1+\frac{4004}{4096}}+1+\frac{113}{256}}=9+\frac{9}{16}+\sqrt{2+\frac{217}{256}}=\left(9+\frac{9}{16}\right)+\left(1+\frac{11}{16}\right)=11+\frac{1}{4}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :Minus a root of a sum of a root of 43 and 272 parts of 4[0]96 | ||
| + | :with a root 50 and 2225 parts of 4096, | ||
| + | :a root of 48 and 3201 parts of 4096, | ||
| + | :and with 7 and 145 parts of 256; | ||
| + | :its total is a root of 28 and 57 parts of 256; | ||
| + | :this root is 5 and 5 parts of 16. | ||
| + | |style="text-align:right;"|פחות שרש מחבור שרש מ"ג ורע"ב חלקי' מתצ"ו<br> | ||
| + | עם שרש מחבור שרש נ' ואלפיים רכ"ה חלקי' מד' אלפי' וצ"ו<br> | ||
| + | ועם שרש מחבור שרש מ"ח וג' אלפי' ר"א חלקי' מד' אלפי' וצ"ו עם <s>שרש מחבור שרש ז'</s> ז' וקמ"ה חלקי' מרנ"ו<br> | ||
| + | אשר סך כלם הוא שרש כ"ח ונ"ז חלקי' מרנ"ו<br> | ||
| + | וזה <sup>השרש</sup> הוא ה' וה' חלקי' מי"ו | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{\sqrt{43+\frac{272}{4{\color{red}{0}}96}}+\sqrt{50+\frac{2225}{4096}}+\sqrt{48+\frac{3201}{4096}}+7+\frac{145}{256}}=\sqrt{28+\frac{57}{256}}=5+\frac{5}{16}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :Minus a root of a sum of a root of 0 and 729 parts of 4096, | ||
| + | :with 0 and 117 parts of 256; | ||
| + | :its total is a root of 225 parts of 256; this root is 15 parts of 16. | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{\sqrt{0+\frac{729}{4096}}+0+\frac{117}{256}}=\sqrt{\frac{225}{256}}=\frac{15}{16}}}</math> | ||
| + | |style="text-align:right;"|‫<ref>13v</ref>פחות שרש מחבור שרש 0 ותשכ"ט חלקי' מד' אלפי' וצ"ו<br> | ||
| + | עם 0 וקי"ז חלקי' מרנ"ו<br> | ||
| + | שיהיה סכומו שרש מרכ"ה חלקי' מרנ"ו אשר זה השרש הוא ט"ו חלקי' מי"ו | ||
| + | |- | ||
| + | | | ||
| + | :Its sum with the first subtractive root, which is 5 and 5 parts of 16, is 6 and a quarter. | ||
| + | |style="text-align:right;"|ו{{#annot:term|154,1208|6RzR}}חבורו עם ה{{#annotend:6RzR}}שרש הראשון מזה {{#annot:term|789,1364|0Fi8}}הפחת{{#annotend:0Fi8}} שהוא ה' <s>וחלק</s> וה' חלקי' מי"ו עולה לסך ו' ורביע | ||
| + | |- | ||
| + | | | ||
| + | :Therefore, 5 remains and this is the result of division of 70 by a root of 4, a root of 9, a root of 16, and a root of 25. | ||
| + | |style="text-align:right;"|אם כן ישאר ה' וככה יעלה לחלוק ע' בשרש ד' ובשר<sup>ש</sup> ט' ובשרש י"ו ובשרש כ"ה | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{70\div\left(\sqrt{4}+\sqrt{9}+\sqrt{16}+\sqrt{25}\right)=\left(11+\frac{1}{4}\right)-\left[\left(5+\frac{5}{16}\right)+\frac{15}{16}\right]=\left(11+\frac{1}{4}\right)-\left(6+\frac{1}{4}\right)=5}}</math> | ||
| + | |- | ||
| + | | | ||
| + | *To divide 70 by the sum of a root of 4, a root of 9, a root of 16, and a root of 25. | ||
| + | |style="text-align:right;"|<big>לחלק</big> ע' בשרש ד' ובשרש ט' ובשרש י"ו ובשרש כ"ה מחוברי' יחד | ||
| + | |- | ||
| + | | | ||
| + | :The result is a root of a sum of a root of 478 and 2112 parts of 4096, | ||
| + | :with a root of 502 and 3033 parts of 4096, | ||
| + | :a root of 542 and 68 parts of 4096, | ||
| + | :and with 23 and [2]21 parts of 256; | ||
| + | |style="text-align:right;"|יעלה שרש מחבור שרש מתע"ח ואלפיים קי"ב חלקי' מד' אלפי' וצ"ו<br> | ||
| + | עם שרש מחבור שרש מתק"ב וג' אלפי' ול"ג חלקי' מד' אלפי' וצ"ו<br> | ||
| + | ועם שרש חבור עם שרש תקמ"ב וס"ח חלקי' מד' אלפי' צ"ו<br> | ||
| + | עם כ"ג ושכ"א חלקי' מרנ"ו | ||
| + | |- | ||
| + | | | ||
| + | :Plus a root of a sum of a root of 1 and 4004 parts of 4096, | ||
| + | :with 1 and 113 parts of 256; | ||
| + | |style="text-align:right;"|ויותר שרש מחבור שרש א' וד' אלפי' וד' חלקי' מד' אלפי' וצ"ו<br> | ||
| + | עם א' וקי"ג חלקי' מרנ"ו | ||
| + | |- | ||
| + | | | ||
| + | :Minus a root of a sum of [a root of] 43 and 272 parts of 4096, | ||
| + | :with a root of 50 and 222[5] parts of 4096, | ||
| + | :a root of 48 and 3201 parts of 4096, | ||
| + | :and with 7 and 145 parts of 256. | ||
| + | |style="text-align:right;"|פחות שרש מחבור מ"ג ורע"ב חלקי' מד' אלפי' וצ"ו<br> | ||
| + | עם שרש מחבור שרש נ' וב' אלפי' רכ"ו חלקי' מד' אלפים וצ"ו<br> | ||
| + | ועם שרש מחבור שרש מ"ח וג' אלפי' ור"א חלקי' מד' אלפי' וצ"ו<br> | ||
| + | עם ז' וקמ"ה חלקי' מרנ"ו | ||
| + | |- | ||
| + | | | ||
| + | :Minus a root of a sum of a root of 0 and 729 parts of 4096 | ||
| + | :with 0 and 117 parts of 256. | ||
| + | |style="text-align:right;"|ופחות שרש מחבור שרש 0 ותשכ"ט חלקי' מד' אלפי' וצ"ו<br> | ||
| + | עם 0 וקי"ז חלקי' מרנ"ו | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle70&\scriptstyle\div\left(\sqrt{4}+\sqrt{9}+\sqrt{16}+\sqrt{25}\right)\\&\scriptstyle=\sqrt{\sqrt{478+\frac{2112}{4096}}+\sqrt{502+\frac{3033}{4096}}+\sqrt{542+\frac{68}{4096}}+23+\frac{{\color{red}{2}}21}{256}}+\sqrt{\sqrt{1+\frac{4004}{4096}}+1+\frac{113}{256}}\\&\scriptstyle-\sqrt{\sqrt{43+\frac{272}{4096}}+\sqrt{50+\frac{222{\color{red}{5}}}{4096}}+\sqrt{48+\frac{3201}{4096}}+7+\frac{145}{256}}-\sqrt{\sqrt{0+\frac{729}{4096}}+0+\frac{117}{256}}\\\end{align}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :When the total is converted to an expressible number, the total result of division is five. | ||
| + | |style="text-align:right;"|ומושב הכל למספר מדובר יעלה אל סך מה שיעלה מן החלוקה שהוא חמשה | ||
| + | |- | ||
| + | |Know that you may encounter many other types of division; they are endless. | ||
| + | |style="text-align:right;"|<big>ודע</big> כי מיני' <sup>רבים</sup> אחרי' מהחלוק אפשר שיזדמנו לך והם בלי תכלית | ||
| + | |- | ||
| + | |But, from the teachings we have presented above, you can deduce a general rule for every multiplication, division, subtraction and addition you may encounter. | ||
| + | |style="text-align:right;"|אבל מן הלמודי' אשר הגדנו למעלה תוכל לתת כלל לכלם לכפל ולחלוק ולמגרעת ולחבור שי<sup>ו</sup>כלו להזדמן לך | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | == <span style=color:Green>Algebra</span> == | ||
| + | |||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | === <span style=color:Green>The Six Canonical Equations</span> === | ||
| + | |||
| + | | | ||
| + | |- | ||
| + | |There are six chapters in the book al-Jīblī al-Mūqabāla: three of them are simple and the others are compound; the third of the three simple can be returned to the first. | ||
| + | |style="width:45%; text-align:right;"|<big>בספר</big> אלג'יבְלֵי אמוגאבאלא יש בו ששה פרקים ומהם שלשה פשוטי' והאחרים הם מורכבי' והשלישי מהג' הפשוטי' אפשר להשיבו אל הראשון | ||
| + | |- | ||
| + | |One can observe these chapters and create many other species that can be returned to this nature or to the similarity of the mentioned chapters, as you can see in the example of the amendments that are set from here on. | ||
| + | |style="text-align:right;"|ועל אלו הפרקי' אפשר להתבונן ולעשות מיני' רבים אחרים אשר בעבור זה ישובו אל הטבע או אל דמיון הפרקי' האמורי' כפי אשר תראה במשל בתקוני' אשר נניח מכאן ולהבא | ||
| + | |- | ||
| + | |By these chapters, with the amendments, it is possible to reach through demonstration to profound concealed subtle calculations, either of arithmetic or of geometry. | ||
| + | |style="text-align:right;"|אשר עם אלו הפרקי' עם התקוני' אפשר להגיע בבאור אל חשבונו' עמוקי' ונסתרי' ודקים בין מה{{#annot:term|365,1637|fTqM}}אריסמיטיקא{{#annotend:fTqM}} ובין מה{{#annot:term|302,1301|zdcI}}גימטריא{{#annotend:zdcI}} | ||
| + | |- | ||
| + | |The chapters and their nature are written below: | ||
| + | |style="text-align:right;"|אלו הכתובי' תחת זה הם הפרקי' וטבעם | ||
| + | |- | ||
| + | | | ||
| + | *The first chapter: a thing equal to a number. | ||
| + | |style="text-align:right;"|הפרק הראשון יבא דבר שוה למספר | ||
| + | |- | ||
| + | | | ||
| + | *The second chapter: a square equal to a number. | ||
| + | |style="text-align:right;"|הפרק השני יבא צינסו שוה למספר | ||
| + | |- | ||
| + | | | ||
| + | *The third chapter: a thing equal to a square. | ||
| + | |style="text-align:right;"|הפרק השלישי יבא דבר שוה לצינסו | ||
| + | |- | ||
| + | | | ||
| + | *The fourth chapter: a square and thing equal to a number. | ||
| + | |style="text-align:right;"|הפרק הרביעי יבא צינסו ודבר שוה למספר | ||
| + | |- | ||
| + | | | ||
| + | *The fifth chapter: a square and number equal to a thing. | ||
| + | |style="text-align:right;"|הפרק החמישי יבא צינסו ומספר שוה לדבר | ||
| + | |- | ||
| + | | | ||
| + | *The sixth chapter: a [thing] and number equal to a square. | ||
| + | |style="text-align:right;"|הפרק הששי יבא צינסו ומספר שוה לצינסו | ||
| + | |- | ||
| + | | | ||
| + | *The nature of the first chapter: when the things are equal to a number. | ||
| + | :<math>\scriptstyle bx=c</math> | ||
| + | |style="text-align:right;"|‫<ref>14r</ref><big>הטבע</big> מן הפרק הראשון הוא זה כאשר הדברי' יהיו שוים אל המספר | ||
| + | |- | ||
| + | | | ||
| + | :The number should be divided by the number of the things and the result is equal to the thing. | ||
| + | :<math>\scriptstyle x=\frac{c}{b}</math> | ||
| + | |style="text-align:right;"|צריך לחלק המספר בכמות הדברים והעולה מהן שוה הדבר | ||
| + | |- | ||
| + | | | ||
| + | :*Suppose, for example, that three things are equal to 12. | ||
| + | ::<math>\scriptstyle3x=12</math> | ||
| + | |style="text-align:right;"|{{#annot:term|898,1896|uKD5}}נניח המשל{{#annotend:uKD5}} ונאמ' כי שלשה דברי' יהיו שוים לי"ב | ||
| + | |- | ||
| + | | | ||
| + | ::Divide the number, which is 12, by the number of the things, which is 4; the result is 4 and this is the thing. | ||
| + | ::<math>\scriptstyle{\color{blue}{x=\frac{12}{3}=4}}</math> | ||
| + | |style="text-align:right;"|חלק המספר שהוא י"ב בכמויות הדברי' שהם ג' ויעלה מהם ד' וככה שוה הדבר | ||
| + | |- | ||
| + | | | ||
| + | ::So, if the thing is 4, 3 things are equal to 12. | ||
| + | ::<math>\scriptstyle{\color{blue}{x=4\longrightarrow3x=12}}</math> | ||
| + | |style="text-align:right;"|אם כן אם הדבר הוא ד' ג' דברי' היטב הם שוים אל י"ב | ||
| + | |- | ||
| + | | | ||
| + | *The nature of the second chapter: when the squares are equal to a number. | ||
| + | :<math>\scriptstyle ax^2=c</math> | ||
| + | |style="text-align:right;"|<big>הטבע</big> מן הפרק השני הוא זה כאשר הצינסי יהיו שוים אל המספר | ||
| + | |- | ||
| + | | | ||
| + | :The number should be divided by the number of the squares and the result is equal to the square. | ||
| + | :<math>\scriptstyle x^2=\frac{c}{a}</math> | ||
| + | |style="text-align:right;"|צריך לחלק המספר בכמויות ה{{#annot:term|687,1313|uHk9}}צינסי{{#annotend:uHk9}} והעולה מזה ככה שוה <s>הדבר</s> הצינסו | ||
| + | |- | ||
| + | | | ||
| + | :The thing is the root of the result, bcause the thing is a root of the square. | ||
| + | :<math>\scriptstyle x=\sqrt{x^2}=\sqrt{\frac{c}{a}}</math> | ||
| + | |style="text-align:right;"|והדבר הוא השרש מאשר יגיע מפני כי הדבר הוא שרש מהצינסו | ||
| + | |- | ||
| + | | | ||
| + | :*Suppose that 2 squares are equal to 32. | ||
| + | ::<math>\scriptstyle2x^2=32</math> | ||
| + | |style="text-align:right;"|נניח כי ב' צינסי יהיו שוים אל ל"ב | ||
| + | |- | ||
| + | | | ||
| + | ::Divide the number, which is 32, by the number of the squares, which is 2; the result is 16 and this is the thing. | ||
| + | ::<math>\scriptstyle{\color{blue}{x^2=\frac{32}{2}=16}}</math> | ||
| + | |style="text-align:right;"|תחלק המספר שהוא ל"ב בכמויות הצינסי שהם ב' ויהיה המגיע י"ו וככה שוה הצינסו | ||
| + | |- | ||
| + | | | ||
| + | ::The thing is its root, i.e. a root of 16, which is 4. | ||
| + | ::<math>\scriptstyle{\color{blue}{x=\sqrt{16}=4}}</math> | ||
| + | |style="text-align:right;"|והדבר הוא שרשו דהיינו שרש מי"ו שהוא ד‫' | ||
| + | |- | ||
| + | | | ||
| + | ::So, if the square is 16, 2 squares are equal to 32. | ||
| + | ::<math>\scriptstyle{\color{blue}{x^2=16\longrightarrow2x^2=32}}</math> | ||
| + | |style="text-align:right;"|ולכן אם הצינסו הוא י"ו ב' צינסי היטב יהיו שוים לל"ב | ||
| + | |- | ||
| + | | | ||
| + | *The nature of the third chapter: when the things are equal to squares. | ||
| + | :<math>\scriptstyle ax^2=bx</math> | ||
| + | |style="text-align:right;"|<big>הטבע</big> מהפרק השלישי הוא זה כאשר הדברי' הם שוים לצינסי | ||
| + | |- | ||
| + | | | ||
| + | :The number of the things should be divided by the number of the squares and the result is a number that is equal to the thing. | ||
| + | :<math>\scriptstyle x=\frac{b}{a}</math> | ||
| + | |style="text-align:right;"|צריך לחלק כמויות הדברי' בכמויות הצינסי והעולה מזה הוא מספר וככה שוה הדבר | ||
| + | |- | ||
| + | | | ||
| + | :*Suppose that 2 squares are equal to 6 things. | ||
| + | ::<math>\scriptstyle2x^2=6x</math> | ||
| + | |style="text-align:right;"|נניח כי ב' צינצי יהיו שוים אל ו' דברים | ||
| + | |- | ||
| + | | | ||
| + | ::Divide the number of the things, which is 6, by the number of the squares, which is 2; the result is 3 and this is the thing. | ||
| + | ::<math>\scriptstyle{\color{blue}{x=\frac{6}{2}=3}}</math> | ||
| + | |style="text-align:right;"|תחלק כמויות הדברי' שהם ו' בכמויות הצינסי שהם ב' ויהיה העולה ג' וככה שוה הדבר | ||
| + | |- | ||
| + | | | ||
| + | ::Since the thing is 3, the square is 9; and since the square is 9, 2 squares are 18. | ||
| + | ::<math>\scriptstyle{\color{blue}{x=3\longrightarrow x^2=9\longrightarrow2x^2=18}}</math> | ||
| + | |style="text-align:right;"|וזה הדבר בהיותו ג' הצינסו יהיה ט' ואם הצינסו הוא ט' א"כ ב' צינסי יהיו י"ח | ||
| + | |- | ||
| + | | | ||
| + | ::When the thing is 3, 6 things are 18, therefore, 2 squares are equal to 6 things | ||
| + | ::<math>\scriptstyle{\color{blue}{x=3\longrightarrow6x=18\longrightarrow2x^2=6x}}</math> | ||
| + | |style="text-align:right;"|ובהיות הדבר ג' ו' דברי' יפה הם שוים י"ח ולכן ב' צינצי יהיו היטב שוים לו' דברי‫' | ||
| + | |- | ||
| + | | | ||
| + | :Know that the third chapter can be returned to the first [chapter] as said above, by dividing the equation by a thing, which is the amendment, as you will see later in the said book | ||
| + | |style="text-align:right;"|<big>ודע</big> כי זה הפרק השלישי אפשר להשיבו אל הראשון כפי האמור למעלה אֵיסְקִיסאנְדּוֹ האַדֵּיקְוואצִיאוֹנֵי הוא התקון בדבר כמו שתראה בהמשך הספר האמור | ||
| + | |- | ||
| + | | | ||
| + | *The nature of the fourth chapter, which is the first of the compound equations, since one quantity is equal to two other different quantities, is when the squares and things are equal to numbers. | ||
| + | :<math>\scriptstyle ax^2+bx=c</math> | ||
| + | |style="text-align:right;"|<big>הטבע</big> מהפרק הרביעי שהוא הראשון מהמורכבי' בעבור כי אחד או כמות אחד יזדמן שיושם שוה לשני כמויות אחרים מתחלפים הוא זה כאשר הצינסי והדברי' יהיו שוים למספרי‫' | ||
| + | |- | ||
| + | | | ||
| + | :<span style=color:Green>'''Normalization''':</span> The whole equation should be divided by the number of the squares. | ||
| + | :<math>\scriptstyle x^2+\frac{b}{a}x=\frac{c}{a}</math> | ||
| + | |style="text-align:right;"|צריך לחלק כל הדיקוואציאוני בכמויות הצינסי | ||
| + | |- | ||
| + | | | ||
| + | :Then, the number of the things should be divided into two equal parts. | ||
| + | |style="text-align:right;"|ואח"כ לחלק כמויות הדברי' לשני חלקי' שוים | ||
| + | |- | ||
| + | | | ||
| + | :Multiply one of these parts, which is its half, by itself. | ||
| + | |style="text-align:right;"|ואחד מאותם החלקי' שהוא חצים תכה בעצמו | ||
| + | |- | ||
| + | | | ||
| + | :Add it to the number. | ||
| + | |style="text-align:right;"|ו{{#annot:term|178,1206|zdWP}}הוסיפם על{{#annotend:zdWP}} כמויות המספר | ||
| + | |- | ||
| + | | | ||
| + | :The root of this sum minus the other half of [the number of] the things is the thing. | ||
| + | :<math>\scriptstyle x=\sqrt{\left(\frac{1}{2}\sdot\frac{b}{a}\right)^2+\frac{c}{a}}-\left(\frac{1}{2}\sdot\frac{b}{a}\right)</math> | ||
| + | |style="text-align:right;"|ושרש זה הסכום פחות החצי האחר מן הדברי' הוא הדבר | ||
| + | |- | ||
| + | | | ||
| + | :*For example, suppose that 2 squares and 20 things are equal to 78 numbers. | ||
| + | ::<math>\scriptstyle2x^2+20x=78</math> | ||
| + | |style="text-align:right;"|והנה המשל נניח כי ב' צינסי וכ' דברי' יהיו שוים אל <s>כ</s> ע"ח דראמי דהיינו מספרי‫' | ||
| + | |- | ||
| + | | | ||
| + | ::<span style=color:Green>'''Normalization''':</span> Now, you should divide the whole equation by the number of the squares. | ||
| + | |style="text-align:right;"|הנך צריך עתה לחלק כל הדיקוואציאוני בכמויות הצינסי | ||
| + | |- | ||
| + | | | ||
| + | ::You get one square and 10 things equal 39. | ||
| + | ::{{#annot:x²+10x=39|710|jJWT}}<math>\scriptstyle x^2+10x=39</math> | ||
| + | |style="text-align:right;"|ויהיה לך צינסו אחד וי' דברי' שוים לל"ט{{#annotend:jJWT}} | ||
| + | |- | ||
| + | | | ||
| + | ::Then, divide the number of the things in half, meaning by 2; each part is 5. | ||
| + | |style="text-align:right;"|אח"כ תחלק כמויות הדברי' לחצי רצוני על ב' ויהיה כל אחד מהחלקי' ה‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Multiply 5 by itself; we get 25. | ||
| + | |style="text-align:right;"|וזה הה' תכפול בעצמו ויהיה לנו כ"ה | ||
| + | |- | ||
| + | | | ||
| + | ::Add the 25 to the number, which is 39; you get 64. | ||
| + | |style="text-align:right;"|והוסיף אלו הכ"ה על המספר שהוא ל"ט ויהיה לך ס"ד | ||
| + | |- | ||
| + | | | ||
| + | ::The root of this sum, meaning the root of 64, minus the other half of [the number of] the things, i.e. minus 5, is the thing, which is 3. | ||
| + | |style="text-align:right;"|ושרש זה הסך רצוני שרש ס"ד פחות החצי האחר מהדברי' הוא הדבר<br> | ||
| + | דהיינו פחות ה' וזה הדבר בהרצות במספר ידוע ‫<ref>14v</ref>יהיה ג‫' | ||
| + | |- | ||
| + | | | ||
| + | ::The reason is that the root of 64 is 8. | ||
| + | |style="text-align:right;"|והסבה היא זאת כי שרש ס"ד הוא ח‫' | ||
| + | |- | ||
| + | | | ||
| + | ::We subtract half [the number of] the things, which is 5, from 8; 3 remains, so the thing is 3. | ||
| + | |style="text-align:right;"|ומזה הח' נוציא החצי מכמ<sup>ו</sup>יות הדברי' שהוא ה' וישאר ג' א"כ הדבר הוא ג‫' | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | ::<math>\scriptstyle{\color{blue}{x=\sqrt{\left(\frac{1}{2}\sdot10\right)^2+39}-\left(\frac{1}{2}\sdot10\right)=\sqrt{5^2+39}-5=\sqrt{25+39}-5=\sqrt{64}-5=8-5}}</math> | ||
| + | |- | ||
| + | | | ||
| + | ::The square is its product by itself, which is 9. | ||
| + | ::<math>\scriptstyle{\color{blue}{x^2=3^2=9}}</math> | ||
| + | |style="text-align:right;"|והצינסו הוא הכאתו בעצמו שהוא ט‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Therefore, when one square is 9 and 10 things, such that the thing is 3, are 30, and they are summed together, they are equal to 39. | ||
| + | ::<math>\scriptstyle{\color{blue}{x^2+10x=9+30=39}}</math> | ||
| + | |style="text-align:right;"|א"כ בהיות הצינסו האחד ט' וי' דברי' כשישוה הדבר ג' יהיו ל' וכשיחוברו יחד יהיו היטב שוים אל ל"ט | ||
| + | |- | ||
| + | | | ||
| + | *The nature of the fifth chapter, meaning the second of the compound equations, is when the squares and number are equal to [things]. | ||
| + | :<math>\scriptstyle ax^2+c=bx</math> | ||
| + | |style="text-align:right;"|<big>הטבע</big> מהפרק החמישי רצוני השני מהמורכבי' הוא זה כאשר הצינסי והמספר יהיו שוים למספר | ||
| + | |- | ||
| + | | | ||
| + | :<span style=color:Green>'''Normalization''':</span> The whole equation should be divided by the number of the squares. | ||
| + | :<math>\scriptstyle x^2+\frac{c}{a}=\frac{b}{a}x</math> | ||
| + | |style="text-align:right;"|צריך לחלק כל האדיקוואציאוני בכמויות הצינצי | ||
| + | |- | ||
| + | | | ||
| + | :Then, [the number of] the things should be divided by two. | ||
| + | |style="text-align:right;"|ואח"כ לחלק הדברי' לשנים | ||
| + | |- | ||
| + | | | ||
| + | :Multiply one of these half, meaning the number of one of these parts, by itself. | ||
| + | |style="text-align:right;"|ואחד מאותם החצאים רצוני הכמות מאחד מאותם החלקי' תכפול בעצמו | ||
| + | |- | ||
| + | | | ||
| + | :Subtract the number from this product. | ||
| + | |style="text-align:right;"|ומאותו הכפל תוציא המספר | ||
| + | |- | ||
| + | | | ||
| + | :Add the root of the remainder to the other half of the number of the things and this is equal to the thing. | ||
| + | :<math>\scriptstyle x=\left(\frac{1}{2}\sdot\frac{b}{a}\right)+\sqrt{\left(\frac{1}{2}\sdot\frac{b}{a}\right)^2-\frac{c}{a}}</math> | ||
| + | |style="text-align:right;"|ושרש הנשאר תוסיף על החצי האחר מכמות הדברי' וככה יהיה שוה הדבר | ||
| + | |- | ||
| + | | | ||
| + | :Or, subtract the root of the remainder from the other half of the number of the things and the result is the value of the thing. | ||
| + | :<math>\scriptstyle x=\left(\frac{1}{2}\sdot\frac{b}{a}\right)-\sqrt{\left(\frac{1}{2}\sdot\frac{b}{a}\right)^2-\frac{c}{a}}</math> | ||
| + | |style="text-align:right;"|או תוציא שרש הנשאר מהחצי האחר מכמות הדברי' וככה יעלה שווי הדבר | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|<big>ודע</big> כי חשבונות מה תצטרך להשיב שיהיה הדבר באופן הראשון רצוני שיהיה החצי מכמות הדברי' ויותר שרש מהנשאר | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|וחשבונות אחרי' מה באופן השני שהוא החצי מכמות הדברי' פחות שרש הנשאר | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ויש אשר אפשר לענות בם בשני האופני‫' | ||
| + | |- | ||
| + | | | ||
| + | :*For example, suppose that 3 squares and 63 numbers are equal to 30 things. | ||
| + | ::<math>\scriptstyle3x^2+63=30x</math> | ||
| + | |style="text-align:right;"|והנה המשל נניח כי ג' צינסי וס"ג מספרי' יהיו שוים לל' דברי‫' | ||
| + | |- | ||
| + | | | ||
| + | ::<span style=color:Green>'''Normalization''':</span> First, you should divide the whole equation by the number of the squares, which is 3. | ||
| + | |style="text-align:right;"|הנך צריך לחלק ראשונה כל האדיקוואציאוני בכמות הצינסי שהוא ג‫' | ||
| + | |- | ||
| + | | | ||
| + | ::{{#annot:x²+21=10x|711|RpEs}}You get one square and 21 numbers equal 10 things. | ||
| + | ::<math>\scriptstyle x^2+21=10x</math> | ||
| + | |style="text-align:right;"|ויהיה לך א' צינסו וכ"א מספרי' שוים לי' דברי‫'{{#annotend:RpEs}} | ||
| + | |- | ||
| + | | | ||
| + | ::Then, divide the number of the things in half; you get each part 5. | ||
| + | |style="text-align:right;"|אח"כ תחלק כמות הדברי' לחצי ויהיה לך כל חלק ה‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Multiply 5 by itself; the result is 25. | ||
| + | |style="text-align:right;"|וזה הה' תכפול בעצמו ועולה כ"ה | ||
| + | |- | ||
| + | | | ||
| + | ::Subtract the number, which is 21, from it; 4 remains. | ||
| + | |style="text-align:right;"|ומהם תוציא המספר שהוא כ"א וישאר ד‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Add the root of 4 to, or subtract from the other half of [the number of] the things; you receive that the thing is 5 plus a root of 4, or 5 minus a root of 4. | ||
| + | |style="text-align:right;"|ושרש ד' תוסיף על החצי האחר מהדברי' או תגרע ויגיע לך כי הדבר יהיה ה' ושרש ד' או ה' פחות שרש ד‫' | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | ::<math>\scriptstyle{\color{blue}{x=\left(\frac{1}{2}\sdot10\right)\pm\sqrt{\left(\frac{1}{2}\sdot10\right)^2-21}=5\pm\sqrt{5^2-21}=5\pm\sqrt{25-21}=5\pm\sqrt{4}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ופעמי' מה תוכל להשיב שהדבר הוא כפי האופן הראשון ולא כשני ופעמי' מה כפי השני ולא כראשון כמו שתראה מכאן ולהבא בחשבונות מה יושמו לזה הקפיטולו | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{x_1=5+\sqrt{4}=5+2=7}}</math> | ||
| + | |style="text-align:right;"|ואם תשיב היות הדבר כפי האופן הראשון שהוא ה' ושרש ד' שהוא ב' יהיה לך היות הדבר ז‫' | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{10x_1=70}}</math> | ||
| + | |style="text-align:right;"|ואם הדבר הוא ז' י' דברי' יהיו ע‫' | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{x_1^2=49}}</math> | ||
| + | |style="text-align:right;"|והצינסו יהיה מ"ט בהיות הדבר ז‫' | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{x_1^2+21=10x}}</math> | ||
| + | |style="text-align:right;"|א"כ א' צינסו עם כ"א יותר יגיע היטב להיות שוה לי' דברים | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{x_2=5-\sqrt{4}=5-2=3}}</math> | ||
| + | |style="text-align:right;"|ואם תענה היות הדבר כפי האופן השני שהוא ה' פחות שרש ד' אשר זה השרש הוא ב' ישאר להיות הדבר ג‫' | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{10x_2=30}}</math> | ||
| + | |style="text-align:right;"|וי' הדברי' יהיו ל‫' | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{x_2^2=9}}</math> | ||
| + | |style="text-align:right;"|והצינסו יהיה ט' בהיות הדבר ג‫' | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|א"כ א' צינסו עם כ"א יותר יהיו היטב שוים אל י' דברים בשני האופני‫' | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|אבל הכרח מענה האופן הראשון עם של השני אי אפשר לראותו במשל הפרק לבד למה יתן להיות הדבר באופן <s>השני</s> <sup>האחד</sup> והשני אבל תראה ההכרח מהתשובות מכאן ולהבא בהמשך הספר האמור | ||
| + | |- | ||
| + | | | ||
| + | *The nature of the sixth chapter, which is the third of the compound equations, is when the things and number are equal to squares. | ||
| + | :<math>\scriptstyle bx+c=ax^2</math> | ||
| + | |style="text-align:right;"|<big>הטבע</big> מהפרק הששי שהוא השלישי המורכב הוא זה כאשר הדברי' והמספרי' יהיו שוים אל הצינסי | ||
| + | |- | ||
| + | | | ||
| + | :<span style=color:Green>'''Normalization''':</span> The whole equation should be divided by the number of the squares. | ||
| + | :<math>\scriptstyle\frac{b}{a}x+\frac{c}{a}=x^2</math> | ||
| + | |style="text-align:right;"|‫<ref>marg.: תמצאהו בראש העלה הרביעי אחר זה</ref>צריך לחלק ‫<ref>15r</ref>כל האדיקוואציאוני בכמות הצינסי | ||
| + | |- | ||
| + | | | ||
| + | :Then, [the number of] the things should be divided by two. | ||
| + | |style="text-align:right;"|ואח"כ לחלק הדברי' לשנים | ||
| + | |- | ||
| + | | | ||
| + | :Multiply one of these half, meaning its number, by itself. | ||
| + | |style="text-align:right;"|ואחד מאלו החצאי' רצוני כמותו תכפול בעצמו | ||
| + | |- | ||
| + | | | ||
| + | :Add this product to the number. | ||
| + | |style="text-align:right;"|והכפל הזה תוסיף על המספרי‫' | ||
| + | |- | ||
| + | | | ||
| + | :The root of the whole sum plus half [the number of] the things is the thing. | ||
| + | :<math>\scriptstyle x=\left(\frac{1}{2}\sdot\frac{b}{a}\right)+\sqrt{\left(\frac{1}{2}\sdot\frac{b}{a}\right)^2+\frac{c}{a}}</math> | ||
| + | |style="text-align:right;"|ושרש כל הסכום ויותר כמות מחצית הדברי' הוא הדבר | ||
| + | |- | ||
| + | | | ||
| + | :*{{#annot:3x+4=x²|712|GUT6}}For example, suppose that 3 things and 4 numbers are equal to 1 square. | ||
| + | ::<math>\scriptstyle3x+4=x^2</math> | ||
| + | |style="text-align:right;"|והנה המשל נניח כי שלשה דברי' וד' דרמי רצוני ד' מספרי' יהיו שוים אל א' צינסו{{#annotend:GUT6}} | ||
| + | |- | ||
| + | | | ||
| + | ::<span style=color:Green>'''Normalization''':</span> You should divide the whole equation by the number of the squares, which is 1; the result is the equation itself. | ||
| + | |style="text-align:right;"|הנך צריך לחלק כל האדיקוואציאוני בכמות הצינסי שהוא א' ויבא <s>זה בעצמו</s> זאת ה{{#annot:term|698|gmyo}}שאלה{{#annotend:gmyo}} בעצמה | ||
| + | |- | ||
| + | | | ||
| + | ::So, divide the number of the things in half; you get 1 and a half. | ||
| + | |style="text-align:right;"|ולכן תחלק כמות הדברי' לחצי ויהיה לך א' וחצי | ||
| + | |- | ||
| + | | | ||
| + | ::Multiply it by itself; the result is 2 and a quarter. | ||
| + | |style="text-align:right;"|תכפלהו על עצמו ויגיע ב' ורביע | ||
| + | |- | ||
| + | | | ||
| + | ::Add it to the number; you get 6 and a quarter. | ||
| + | |style="text-align:right;"|וזה תוסיף על המספר ויהיה לך ו' ורביע | ||
| + | |- | ||
| + | | | ||
| + | ::The root of 6 and a quarter plus the other half of the number of the things is the thing. | ||
| + | |style="text-align:right;"|ושרש ו' ורביע ויותר החצי האחר מכמות הדברי' הוא הדבר | ||
| + | |- | ||
| + | | | ||
| + | ::The root of 6 and a quarter is 2 and a half. | ||
| + | |style="text-align:right;"|ושרש ו' ורביע הוא ב' וחצי | ||
| + | |- | ||
| + | | | ||
| + | ::When added to half the number of the things, which is 1 and a half; the result is 4. | ||
| + | |style="text-align:right;"|ובחברו על מחצית כמות הדברי' שהוא א' וחצי עושה ד‫' | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | ::<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle x&\scriptstyle=\left(\frac{1}{2}\sdot3\right)+\sqrt{\left(\frac{1}{2}\sdot3\right)^2+4}=\left(1+\frac{1}{2}\right)+\sqrt{\left(1+\frac{1}{2}\right)^2+4}=\left(1+\frac{1}{2}\right)+\sqrt{\left(2+\frac{1}{4}\right)+4}\\&\scriptstyle=\left(1+\frac{1}{2}\right)+\sqrt{6+\frac{1}{4}}=\left(1+\frac{1}{2}\right)+\left(2+\frac{1}{2}\right)=4\\\end{align}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{x^2=16}}</math> | ||
| + | |style="text-align:right;"|א"כ אם הדבר הוא ד' והצינסו יהיה הכאתו בעצמו והוא י"ו | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{3x=12}}</math> | ||
| + | |style="text-align:right;"|ובהיות הדבר ד' ג' דברי' יהיו י"ב | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{3x+4=16=x^2}}</math> | ||
| + | |style="text-align:right;"|א"כ ג' דברי' וד' יותר היטב יהיו שוים לא' צינסו שהוא י"ו | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|בכאן יראה התחכמות האדיקוואציאוני הם ה{{#annot:term|698|zCuO}}שאלות{{#annotend:zCuO}} לפי דעתי | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|<big>דע</big> כי לעולם אם יזדמן לך איזו שאלה מורכבת שתחלק לעולם כל השאלה בכמו שהוא כמות הצינסו ו{{#annot:term|970,1431|RVwp}}להשיבה{{#annotend:RVwp}} לעולם לא' צינסו כאשר הראית בשאלות מהג' פרקי' המורכבי' האמורי' למעלה | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|וכן בדומה לזה תמשיך הענין בכל אחת מהשאלות המורכבות לחלק לעולם כל השאלה בכמות אשר באחדותו יש לו מהות גדול מאחדות מה מכמויות שיהיו <s>באלו</s> בשאלה ההיא | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ואח"כ תמשיך טבע הפרק כפי מה שהראית ושעתיד להראות מכאן ולהבא | ||
| + | |- | ||
| + | | | ||
| + | ==== <span style=color:Green>Geometric Illustrations of the Three Compound Equations</span> ==== | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|בכאן יראה {{#annot:term|303,1510|NU0p}}צורת ה{{#annotend:NU0p}}ג' פרקים המורכבים | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|<big>עוד</big> רצוני להראותך טבע אלו הג' פרקי' המורכבים בצורת כל אחד לבדו | ||
| + | |- | ||
| + | | | ||
| + | :*<math>\scriptstyle x^2+10x=39</math> | ||
| + | |style="text-align:right;"|איך א' צינסו וי' דברי' יבא {{#annot:term|198,1898|yJsr}}מופת ש{{#annotend:yJsr}}הם שוים אל ל"ט | ||
| + | |- | ||
| + | | | ||
| + | :Since the thing that we denominated is the root of the square, the square is a quadrilateral right-angled equilateral surface. | ||
| + | |style="text-align:right;"|בהיות כי הדבר אשר נקבנו בשם הוא {{#annot:term|133,1262|FM7v}}שרש הצינסו{{#annotend:FM7v}} ולכן יהיה הצינסו {{#annot:term|814,1310|qKhs}}שטח{{#annotend:qKhs}} אחד {{#annot:term|305,2560|wYJE}}מרובע נצב הזויות שוה הצלעות{{#annotend:wYJE}} <s>וישרים</s> | ||
| + | |- | ||
| + | | | ||
| + | :[[File:Dardi 1.png|thumb|170px|left]] | ||
| + | |[[File:דארדי 1.png|thumb|170px|right]] | ||
| + | |- | ||
| + | | | ||
| + | :We draw its shape as an equilateral right-angled quadrilateral. We say that this is the square and it is surface AB. | ||
| + | :<math>\scriptstyle{\color{blue}{AB=x^2}}</math> | ||
| + | |style="text-align:right;"|ולכן {{#annot:term|819,2567|MU8F}}נצייר{{#annotend:MU8F}} צו<sup>ר</sup>ת זה {{#annot:term|305,2560|d9eX}}מרובע אחד שוה הצלעות והזיות נצבות{{#annotend:d9eX}} ונאמר כי זה ה{{#annot:term|305,1263|j1oW}}מרובע{{#annotend:j1oW}} הוא הצינסו והוא השטח שעליו א"ב | ||
| + | |- | ||
| + | | | ||
| + | :Since the thing [<math>\scriptstyle{\color{blue}{x}}</math>] is the root of the square, it is the sides of the stated square. | ||
| + | |style="text-align:right;"|ומפני כי הדבר הוא שרש הצינסו יהיה צלעו' למרובע האמור | ||
| + | |- | ||
| + | | | ||
| + | :As 10 things are added to the square, we divide these 10 things into four parts, each part is 2 things and a half. | ||
| + | :<math>\scriptstyle{\color{blue}{10x\div4=\left(2+\frac{1}{2}\right)x}}</math> | ||
| + | |style="text-align:right;"|ובהיות כי {{#annot:term|178,1213|Or7I}}נוסף על{{#annotend:Or7I}} הצינסו י' דברים אנו נחלק אלו הי' דברים בד' חלקים ויגיע לכל חלק ב' דברים וחצי | ||
| + | |- | ||
| + | | | ||
| + | :Since the thing is the sides of the square, we attach each of these four parts to the square - each part to one side of the square. | ||
| + | |style="text-align:right;"|ובהיות כי הדבר הוא צלעות הצינסו אנחנו {{#annot:term|2052,2049|yNEQ}}נדביק{{#annotend:yNEQ}} כל אחד מאלו הד' חלקי' ‫<ref>15v</ref>אל הצינסו וכל חלק לבדו לצלעו מהצינסו | ||
| + | |- | ||
| + | | | ||
| + | :We get four surfaces, the breadth of each of which is 2 and a half, their length is as the length of the sides of the square [<math>\scriptstyle{\color{blue}{x}}</math>], and the area of each is GD. | ||
| + | |style="text-align:right;"|ויהיו לנו ד' {{#annot:term|814,1310|fIHT}}שטחי'{{#annotend:fIHT}} כל אחד מהם יהיה {{#annot:term|317,1488|P8YW}}רחבו{{#annotend:P8YW}} ב' וחצי וארכו כאורך צלעות הצינסו ו{{#annot:term|816,1310|s6dl}}שטח{{#annotend:s6dl}} כל אחד מהם עליו ג"ד | ||
| + | |- | ||
| + | | | ||
| + | :On each corner of the square there is an equilateral right-angled quadrilateral, whose breadth is 2 and a half, as the breadth of the things, and this breadth, or say length, multiplied by itself yields 6 and a quarter; so its area is 6 and a quarter and it is HW and its side is WZ. | ||
| + | :<math>\scriptstyle{\color{blue}{WZ=2+\frac{1}{2}}}</math> | ||
| + | :<math>\scriptstyle{\color{blue}{HW=\left(2+\frac{1}{2}\right)^2=6+\frac{1}{4}}}</math> | ||
| + | |style="text-align:right;"|ולכל אחד מזויות הצינסו יהיה עליו מרובע אחד שוה הצלעות והזיות נצבות<br> | ||
| + | ויהיה רחבו בצלעותיו כ{{#annot:term|317,1488|ytPj}}רחב ה{{#annotend:ytPj}}דברי' שהוא ב' וחצי<br> | ||
| + | וזה הרחב או אמור הארך מוכה בעצמו יעשה ו' ורביע<br> | ||
| + | א"כ {{#annot:term|816,1310|Q9z1}}שטחו{{#annotend:Q9z1}} יהיה ו' ורביע והוא ה"ו<br> | ||
| + | וצלעו יהיה ו"ז | ||
| + | |- | ||
| + | | | ||
| + | :All these four stated equilateral right-angled surfaces are equal to the breadth of the things in their length and breadth and the sum of their areas is 25. | ||
| + | |style="text-align:right;"|וכל אלו הד' {{#annot:term|305,2560|vsui}}שטחי' שוי הצלעות והזויות נצבות{{#annotend:vsui}} כאמור והם באורך וברחב שוים לרחב הדברי' ושטחיהם יחד <s>שוים</s> <sup>עולים</sup> לסכום כ"ה | ||
| + | |- | ||
| + | | | ||
| + | :Their sides WZ are 2 and a half. | ||
| + | :<math>\scriptstyle{\color{blue}{WZ=2+\frac{1}{2}}}</math> | ||
| + | |style="text-align:right;"|וצלעותיהם ו"ז והוא ב' וחצי | ||
| + | |- | ||
| + | | | ||
| + | :So, we have now a square surface that comprises the square plus the ten things. | ||
| + | :<math>\scriptstyle{\color{blue}{\left[AB+4GD\right]=x^2+10x}}</math> | ||
| + | |style="text-align:right;"|וא"כ יהיה לנו עתה מרובע אחד המחזיק הצינסו ועשרת הדברי‫' | ||
| + | |- | ||
| + | | | ||
| + | :In addition, the area of the four surfaces that are on the corners of the square and are contained in the width of the things is 25 according to what is said and demonstrated. | ||
| + | :<math>\scriptstyle{\color{blue}{\left[4HW\right]=25}}</math> | ||
| + | |style="text-align:right;"|וג"כ אלו הד' <s>ש</s> שטחים שהם חוץ מהזויות מהצינסו {{#annot:term|964,2564|i2ia}}המוחזקי' ב{{#annotend:i2ia}}{{#annot:term|317,2565|4CJI}}מרחב ה{{#annotend:4CJI}}דברי' אשר שטחיהם הוא כ"ה כפי האמור וכמו שהראה | ||
| + | |- | ||
| + | | | ||
| + | :The area of the square with the area of the things is 39. | ||
| + | :<math>\scriptstyle{\color{blue}{x^2+10x=39}}</math> | ||
| + | |style="text-align:right;"|ושטח הצינסו יהיה עם שטח הדברי' ל"ט | ||
| + | |- | ||
| + | | | ||
| + | :Summed with 25, which is the area of the four equilateral quadrilaterals, it yields 64. | ||
| + | :<math>\scriptstyle{\color{blue}{39+25=64}}</math> | ||
| + | |style="text-align:right;"|ומחובר עם כ"ה שהוא שטח הד' {{#annot:term|305,1863|Eq9Q}}מרובעי' השוי הצלעות{{#annotend:Eq9Q}} עושה ס"ד | ||
| + | |- | ||
| + | | | ||
| + | :Therefore, we have quadrilateral CT that comprises all these surfaces and its area is 64, which is equilateral and equiangular [= a square]. | ||
| + | :<math>\scriptstyle{\color{blue}{CT=64}}</math> | ||
| + | |style="text-align:right;"|א"כ יהיה לנו מרובע אחד אשר יחזיק כל אלו השטחי' ושטחו יהיה ס"ד עליו ח"ט ויהיה שוה הצלעות והד' זויות | ||
| + | |- | ||
| + | | | ||
| + | :So, its side is a root of 64. | ||
| + | |style="text-align:right;"|ולכן צלעו יהיה שרש ס"ד | ||
| + | |- | ||
| + | | | ||
| + | :The side of the square [<math>\scriptstyle{\color{blue}{AB=x^2}}</math>] is smaller by 5 [<math>\scriptstyle{\color{blue}{\sqrt{64}-5}}</math>]. | ||
| + | |style="text-align:right;"|וצלע הסינסו יהיה ה' פחות | ||
| + | |- | ||
| + | | | ||
| + | :Because the things that are above and below, or the width of the squares on the corners, are 2 and a half. | ||
| + | |style="text-align:right;"|מפני כי הדברי' אשר ממעל עם אשר מתחת או ג"כ ה{{#annot:term|305,1263|bhc1}}מרובעי'{{#annotend:bhc1}} מהזויות יש להם מ{{#annot:term|317,2565|jOGZ}}מרחב{{#annotend:jOGZ}} ב' וחצי | ||
| + | |- | ||
| + | | | ||
| + | :2 and a half above summed with 2 and a half below yields 5. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(2+\frac{1}{2}\right)+\left(2+\frac{1}{2}\right)=5}}</math> | ||
| + | |style="text-align:right;"|והנה כי ב' וחצי ממעל וב' וחצי מתחת מחוברי' יחד עושה ה‫' | ||
| + | |- | ||
| + | | | ||
| + | :Hence, the side of the square [<math>\scriptstyle{\color{blue}{AB=x^2}}</math>] is smaller by 5 than the side of the square that comprises all these surfaces [= CT]. | ||
| + | |style="text-align:right;"|אם כן יהיה צלע הצינסו ה' פחות מצלע המרובע המחזיק כל אלו השטחי‫' | ||
| + | |- | ||
| + | | | ||
| + | :As you saw it regarding the width of things above and below, you can see it regarding the width of the sides. | ||
| + | |style="text-align:right;"|וכמו שראית זה מפני {{#annot:term|317,1488|gKan}}רוחב ה{{#annotend:gKan}}דברי' ממעל ומתחת וכדומה לזה תוכל לראות ברוחב הדברי' מהצלעות | ||
| + | |- | ||
| + | | | ||
| + | :Since each side, meaning the sides of the square [<math>\scriptstyle{\color{blue}{AB=x^2}}</math>], are equal to each other. | ||
| + | |style="text-align:right;"|מפני כי כל צלע שוה רצוני צלעות הצינסו הם שוים זה לזה | ||
| + | |- | ||
| + | | | ||
| + | :The sides of the great square [CT] are equal to each other. | ||
| + | |style="text-align:right;"|וצלעות המרובע הגדול שוים זה לזה | ||
| + | |- | ||
| + | | | ||
| + | :So, the thing, which is the side of the square [<math>\scriptstyle{\color{blue}{AB=x^2}}</math>] and is a root of the square, is a root of 64 minus 5. | ||
| + | :<math>\scriptstyle{\color{blue}{x=\sqrt{64}-5}}</math> | ||
| + | |style="text-align:right;"|א"כ יהיה הדבר שהוא {{#annot:term|133,1464|03nK}}צלע הצינסו{{#annotend:03nK}} שהוא שרש הצינסו שרש ס"ד פחות ה‫' | ||
| + | |- | ||
| + | | | ||
| + | :This root is 3 as an expressible number. | ||
| + | :<math>\scriptstyle{\color{blue}{x=3}}</math> | ||
| + | |style="text-align:right;"|וזה השרש במספר מדובר הוא ג‫' | ||
| + | |- | ||
| + | | | ||
| + | :If the thing, which is a root of the square, is 3, the square is its product by itself, which is 9, and 9 is the area of the square [AB]. | ||
| + | :<math>\scriptstyle{\color{blue}{x^2=3^2=9}}</math> | ||
| + | |style="text-align:right;"|ואם הדבר שהוא שרש הצינסו יהיה ג‫'<br> | ||
| + | הצינסו יהיה הכאתו בעצמו שהוא ט' וזה הט' הוא שטח הצינסו | ||
| + | |- | ||
| + | |I want to show you this in a different way: | ||
| + | |style="text-align:right;"|<big>עוד</big> רצוני להראותך זה באופן אחר | ||
| + | |- | ||
| + | | | ||
| + | *When we say in a similar way that one square and ten things, or say its ten roots, are equal to 39. | ||
| + | :<math>\scriptstyle x^2+10x=39</math> | ||
| + | |style="text-align:right;"|באמרנו בדומה לזה שצינסו אחד ועשרה דברים או אמור עשרה שרשיו הם שוים לל"ט | ||
| + | |- | ||
| + | | | ||
| + | :[[File:Dardi 2.png|thumb|140px|left]] | ||
| + | |[[File:דארדי 2.png|thumb|140px|right]] | ||
| + | |- | ||
| + | | | ||
| + | :We draw now an equilateral equiangular quadrilateral as the square [<math>\scriptstyle{\color{blue}{x^2}}</math>] and write BG on this surface. | ||
| + | :<math>\scriptstyle{\color{blue}{BG=x^2}}</math> | ||
| + | |style="text-align:right;"|אנחנו נצייר עתה {{#annot:term|305,1863|tWbu}}מרובע אחד שוה הצלעות מד' זויות{{#annotend:tWbu}} בעבור הצינסו ועל השטח רשמנו ב"ג | ||
| + | |- | ||
| + | | | ||
| + | :We divide the ten things into two parts; each part we receive is 5 roots of the square, or say 5 things. | ||
| + | :<math>\scriptstyle{\color{blue}{10x\div2=5x}}</math> | ||
| + | |style="text-align:right;"|ועשרת הדברים אנו מחלקים לב' חלקים ויהיה לנו כי כל חלק יהיה ה' שרשים מהצינסו או אמור ה' דברים | ||
| + | |- | ||
| + | | | ||
| + | :We attach each part to the side of the square, as you see drawn here in this figure. | ||
| + | |style="text-align:right;"|אשר אנחנו {{#annot:term|2052,2049|8VeD}}נדביק{{#annotend:8VeD}} כל חלק אל צלע הצינסו כפי אשר תראה {{#annot:term|819,2566|9Ak5}}מצוייר{{#annotend:9Ak5}} פה ב{{#annot:term|303,1510|FkFL}}זאת הצורה{{#annotend:FkFL}} | ||
| + | |- | ||
| + | | | ||
| + | :We get that these two parts are attached to both sides of the square, and these sides form one corner. The surface of each 5 things is DH. | ||
| + | |style="text-align:right;"|ויהיה לנו שני אלו החלקים {{#annot:term|2053,2048|lkPU}}מדובקים ל{{#annotend:lkPU}}שני צדדי הצינסו אשר אלו הצדדים מחזיקים זוית אחד ושטח כל אחד מאלו הה' דברים יהיה ד"ה | ||
| + | |- | ||
| + | | | ||
| + | :Now, you receive that these two surfaces consist of two sides of an equilateral right-angled quadrilateral, and since its sides are 5, the area of its surface, which is WZ, is 25. | ||
| + | :<math>\scriptstyle{\color{blue}{WZ=25}}</math> | ||
| + | |style="text-align:right;"|עתה יהיה לך שאלו השני שטחים יחזיקו ב' צלעות מ{{#annot:term|305,2560|HdgB}}מרובע אחד שוה הצלעות נצב הזויות{{#annotend:HdgB}} ובהיות צלעותיו ה' יהיה שטחו אשר עליו ו"ז כ"ה | ||
| + | |- | ||
| + | | | ||
| + | :We get that the area of the square plus the area of the things equals 39, because we said that one square plus 10 things equals 39. | ||
| + | :<math>\scriptstyle{\color{blue}{\left[BG+2DH\right]=x^2+10x=39}}</math> | ||
| + | |style="text-align:right;"|ויש לנו שטח הצינסו ושטח הדברים היותם שוים אל ל"ט<br> | ||
| + | מפני כי אמרנו צינסו אחד וי' דברים הם שוים אל ל"ט | ||
| + | |- | ||
| + | | | ||
| + | :So, we receive that the area of WZ, which is 25, summed with 39, equals 64. | ||
| + | :<math>\scriptstyle{\color{blue}{\left[BG+2DH\right]+WZ=39+25=64}}</math> | ||
| + | |style="text-align:right;"|ויהיה לנו השטח שהוא כ"ה אשר עליו ו"ז מחובר עם ל"ט יעלו לסך ס"ד | ||
| + | |- | ||
| + | | | ||
| + | :These four surfaces generate the equilateral right-angled surface CT, whose area is 64. | ||
| + | :<math>\scriptstyle{\color{blue}{\left[BG+2DH+WZ\right]=CT=64}}</math> | ||
| + | |style="text-align:right;"|ואלו הד' שטחים יהיו מחזיקים ב{{#annot:term|305,2560|Jhqq}}שטח אחד שוה הצלעות ונצב הזויות{{#annotend:Jhqq}} אשר שטחו הוא ס"ד ועליו ח"ט | ||
| + | |- | ||
| + | | | ||
| + | :Its side is a root of 64. | ||
| + | |style="text-align:right;"|וצלעו יהיה שרש ס"ד | ||
| + | |- | ||
| + | | | ||
| + | :The side of the square [<math>\scriptstyle{\color{blue}{x^2}}</math>], which is the thing, is smaller by 5. | ||
| + | :<math>\scriptstyle{\color{blue}{x=\sqrt{64}-5}}</math> | ||
| + | |style="text-align:right;"|וצלע הצינסו שהוא הדבר יהיה ה' פחות | ||
| + | |- | ||
| + | | | ||
| + | :We get that the thing is a root of 64, which is 8, when 5 that is smaller than 8 is subtracted from it; 3 remains. | ||
| + | :<math>\scriptstyle{\color{blue}{x=\sqrt{64}-5=8-5=3}}</math> | ||
| + | |style="text-align:right;"|אם כן יהיה לנו שהדבר הוא שרש ס"ד שהוא ח' ו{{#annot:term|181,2562|sbL4}}הוצא מהם{{#annotend:sbL4}} ה' שהוא פחות מח' וישאר ג‫' | ||
| + | |- | ||
| + | | | ||
| + | :Therefore, when the thing is 3, the square, meaning surface BG, is 9. | ||
| + | :<math>\scriptstyle{\color{blue}{BG=x^2=9}}</math> | ||
| + | |style="text-align:right;"|א"כ בהיות הדבר ג' הצינסו יהיה ט' רצוני שטחו אשר עליו ב"ג | ||
| + | |- | ||
| + | | | ||
| + | :The area of each part DH of the things is 15. | ||
| + | :<math>\scriptstyle{\color{blue}{DH=5x=15}}</math> | ||
| + | |style="text-align:right;"|ושטח כל אחד מן החלקים מהדברים שעליהם ד"ה יהיו ט"ו | ||
| + | |- | ||
| + | | | ||
| + | :Thus, one square plus ten things is indeed equal to 39 according to both stated ways illustrated in the figures. | ||
| + | |style="text-align:right;"|א"כ היטב יהיה צינסו אחד ועשרה דברים מראה להיות שוים אל ל"ט מכל אחד משני האופנים האמורים מראים {{#annot:term|303,1510|aHl6}}בצורה{{#annotend:aHl6}} | ||
| + | |- | ||
| + | | | ||
| + | :*<math>\scriptstyle x^2+21=10x</math> | ||
| + | |style="text-align:right;"|בפרק השני המורכב הונח צינסו אחד וכ"א יותר שוה לי' שרשים או אמור לי' דברים | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|וזה הפרק אנחנו מראים מהותו מזאת הצורה | ||
| + | |- | ||
| + | | | ||
| + | :[[File:Dardi 3.png|thumb|220px|left]] | ||
| + | |[[File:דארדי 3.png|thumb|220px|right]] | ||
| + | |- | ||
| + | | | ||
| + | :First, we start with the square that is an equilateral right-angled quadrilateral; we write AB on its surface. | ||
| + | :<math>\scriptstyle{\color{blue}{AB=x^2}}</math> | ||
| + | |style="text-align:right;"|אשר אנחנו מתחילי' ראשונה שיהיה הצינסו {{#annot:term|305,2560|DrKd}}המרובע שוה הצלעות ונצב הזויות{{#annotend:DrKd}} אשר על שטחו רשמנו א"ב | ||
| + | |- | ||
| + | | | ||
| + | :Each of its sides is equal to GD. | ||
| + | |style="text-align:right;"|וכל אחד מצלעיו יהיה שוה לג"ד | ||
| + | |- | ||
| + | | | ||
| + | :We attach surface BZ, which has an area of 21, to side BW. | ||
| + | :<math>\scriptstyle{\color{blue}{BZ=21}}</math> | ||
| + | |style="text-align:right;"|ונוסיף לצלע ב"ו שטח אחד מכ"א אשר עליו ב"ז | ||
| + | |- | ||
| + | | | ||
| + | :So, the area of the number and the square is ten roots of the square. | ||
| + | :<math>\scriptstyle{\color{blue}{\left[DZ\right]=10x}}</math> | ||
| + | |style="text-align:right;"|א"כ אורך השטח מהמספר והצינסו יהיו עשרה שרשים מהצינסו | ||
| + | |- | ||
| + | | | ||
| + | :Since the width of each of these surfaces, which is GD and BW, is as the root of the square. | ||
| + | :<math>\scriptstyle{\color{blue}{GD=BW=x}}</math> | ||
| + | |style="text-align:right;"|מפני כי כל אחד מאלו השטחים יהיה {{#annot:term|317,2565|nsj0}}מרחבם{{#annotend:nsj0}} כשרש הצינסו שהוא ג"ד ב"ו | ||
| + | |- | ||
| + | | | ||
| + | :And the length of both surfaces, which is line GZ, is ten. | ||
| + | :<math>\scriptstyle{\color{blue}{GZ=10}}</math> | ||
| + | |style="text-align:right;"|ואורך שני אלו השטחים הוא כאורך עשרה שרשים והוא קו ג"ז | ||
| + | |- | ||
| + | | | ||
| + | :Divide this length in half and draw line TK from it, so that it is equal to line TZ. | ||
| + | :<math>\scriptstyle{\color{blue}{TK=TZ}}</math> | ||
| + | |style="text-align:right;"|וזה האורך {{#annot:term|786|S7Kl}}תחלק לחצי{{#annotend:S7Kl}} ו{{#annot:term|822,2061|zKyQ}}תמשיך קו אחד עליו{{#annotend:zKyQ}} ט"כ ויהיה שוה לקו ט"ז | ||
| + | |- | ||
| + | | | ||
| + | :The length of this line is then 5. | ||
| + | :<math>\scriptstyle{\color{blue}{TK=\frac{1}{2}\sdot10=5}}</math> | ||
| + | |style="text-align:right;"|ויהיה זה הקו אם כן ארכו ה‫' | ||
| + | |- | ||
| + | | | ||
| + | :Because the length of GZ is ten. | ||
| + | :<math>\scriptstyle{\color{blue}{GZ=10}}</math> | ||
| + | |style="text-align:right;"|מפני כי ג"ז ארכו עשרה | ||
| + | |- | ||
| + | | | ||
| + | :GT is equal to TZ, so each of these lines is 5. | ||
| + | :<math>\scriptstyle{\color{blue}{GT=TZ=\frac{1}{2}\sdot10=5}}</math> | ||
| + | |style="text-align:right;"|וג"ט הוא שוה לט"ז א"כ כל אחד מאלו הקוים יהיה ה‫' | ||
| + | |- | ||
| + | | | ||
| + | :Likewise, DC and CQ are equal to KL. | ||
| + | :<math>\scriptstyle{\color{blue}{DC=CQ=KL}}</math> | ||
| + | |style="text-align:right;"|וכדומה לזה יהיה ד"ח ח"ק אשר הוא שוה לכ"ל | ||
| + | |- | ||
| + | | | ||
| + | :Hence, TK, KL, LZ, ZT are four equal sides, each is 5. | ||
| + | :<math>\scriptstyle{\color{blue}{TK=KL=LZ=ZT=5}}</math> | ||
| + | |style="text-align:right;"|אם כן ט"כ וכ"ל ול"ז וז"ט הם ד' צלעות שוים וכל אחד יהיה ה‫' | ||
| + | |- | ||
| + | | | ||
| + | :So, the square surface KZ is 25. | ||
| + | :<math>\scriptstyle{\color{blue}{KZ=25}}</math> | ||
| + | |style="text-align:right;"|א"כ שטח זה המרובע שעליו כ"ז יהיה כ"ה | ||
| + | |- | ||
| + | | | ||
| + | :Line CK is equal to line WT, because TC is equal to WB and TK is equal to GT. | ||
| + | :<math>\scriptstyle{\color{blue}{TC=WB;\; TK=GT\longrightarrow CK=WT}}</math> | ||
| + | |style="text-align:right;"|וקו ח"כ שוה לקו ו"ט<br> | ||
| + | מפני כי ט"ח הוא שוה לו"ב וט"כ הוא שוה לג"ט | ||
| + | |- | ||
| + | | | ||
| + | :Draw a line from C to N, equal to GW. | ||
| + | :<math>\scriptstyle{\color{blue}{CN=GW}}</math> | ||
| + | |style="text-align:right;"|{{#annot:to draw a line|819,1575|omwB}}תגיע קו אחד מ{{#annotend:omwB}}ח"נ שוה לג"ו | ||
| + | |- | ||
| + | | | ||
| + | :And from N to M, equal to WT. | ||
| + | :<math>\scriptstyle{\color{blue}{NM=WT}}</math> | ||
| + | |style="text-align:right;"|ומנ' אל מ' שוה לו"ט | ||
| + | |- | ||
| + | | | ||
| + | :You get MNQ equal to TW or to BC. | ||
| + | :<math>\scriptstyle{\color{blue}{NQ=TW=BC}}</math> | ||
| + | |style="text-align:right;"|ויהיה לך מנ"ק שוה לט"ו או שוה לב"ח | ||
| + | |- | ||
| + | | | ||
| + | :So, you have an equilateral equiangular quadrilateral [= LN], whose sides are NQ, QL, LM, MN. | ||
| + | :<math>\scriptstyle{\color{blue}{NQ=QL=LM=MN}}</math> | ||
| + | |style="text-align:right;"|א"כ יהיה לך מרובע אחד שוה הצלעות נצב הזויות אשר צלעותיו יהיו נ"ק ק"ל ל"מ מ"נ | ||
| + | |- | ||
| + | | | ||
| + | :Surface NK is equal to surface CW. | ||
| + | :<math>\scriptstyle{\color{blue}{NK=CW}}</math> | ||
| + | |style="text-align:right;"|ושטח נ"כ יהיה שוה לשטח ח"ו | ||
| + | |- | ||
| + | | | ||
| + | :Surface BZ, or say WQ, which is the surface added to the square, is 21. | ||
| + | :<math>\scriptstyle{\color{blue}{BZ=WQ=21}}</math> | ||
| + | |style="text-align:right;"|ושטח ב"ז או אמור ו"ק הוא יהיה כ"א שהוא השטח שנוסף על הצינסו | ||
| + | |- | ||
| + | | | ||
| + | :So, surface TQ plus surface KN are 21, because we leave surface WC and take KN that is equal to WC. | ||
| + | :<math>\scriptstyle{\color{blue}{KN=WC\longrightarrow TQ+KN=21}}</math> | ||
| + | |style="text-align:right;"|א"כ שטח ט"ק ושטח כ"נ יהיו כ"א<br> | ||
| + | מפני כי אנחנו עוזבים שטח ו"ח ולוקחים כ"נ שהוא שוה לו"ח | ||
| + | |- | ||
| + | | | ||
| + | :Therefore, the square surface LN that is equilateral remains 4 and its side is a root of 4. | ||
| + | :<math>\scriptstyle{\color{blue}{LN=4}}</math> | ||
| + | |style="text-align:right;"|א"כ ישאר השטח המרובע שהוא ל"נ שוה הצלעות היותו ד' וצלעו יהיה שרש ד‫' | ||
| + | |- | ||
| + | | | ||
| + | :Because, surface KZ is 25 | ||
| + | :<math>\scriptstyle{\color{blue}{KZ=25}}</math> | ||
| + | |style="text-align:right;"|מפני כי שטח כ"ז הוא כ"ה | ||
| + | |- | ||
| + | | | ||
| + | :And surfaces CZ plus KN are 21 as shown. | ||
| + | :<math>\scriptstyle{\color{blue}{CZ+KN=21}}</math> | ||
| + | |style="text-align:right;"|ושני שטחי ח"ז וכ"נ יהיו כ"א כאשר הראה | ||
| + | |- | ||
| + | | | ||
| + | :Therefore, when QL, which is a root of 4, is subtracted from ZL, which is 5, QZ remains 5 minus a root of 4 and QZ is equal to the side, or say to the root of the square. | ||
| + | :<math>\scriptstyle{\color{blue}{x=QZ=ZL-QL=5-\sqrt{4}}}</math> | ||
| + | |style="text-align:right;"|א"כ ז"ל שהוא ה' {{#annot:term|155,2070|vyPV}}בגרוע ממנו{{#annotend:vyPV}} ק"ל שהוא שרש ד' ישאר ק"ז ה' פחות שרש ד' וק"ז הוא שוה לצלע או אמור אל שרש הצינסו | ||
| + | |- | ||
| + | | | ||
| + | :So, we get that the side of the square, or say its root that is denominated a thing, is 5 minus a root of 4 and when it is converted into an expressible number, it is 3. | ||
| + | :<math>\scriptstyle{\color{blue}{x=\sqrt{x^2}=5-\sqrt{4}=3}}</math> | ||
| + | |style="text-align:right;"|א"כ יהיה לנו שיהיה צלע הצינסו או אמור שרשו אשר ינקב בשם דבר היותו ה' פחות שרש ד‫'<br> | ||
| + | וכש{{#annot:term|1071,2559|b0jH}}הושב אל{{#annotend:b0jH}} מספר מדובר יהיה ג‫' | ||
| + | |- | ||
| + | | | ||
| + | :The square is its product by itself, which is 9. | ||
| + | :<math>\scriptstyle{\color{blue}{x^2=3^2=9}}</math> | ||
| + | |style="text-align:right;"|והצינסו יהיה הכאתו בעצמו שהוא ט‫' | ||
| + | |- | ||
| + | | | ||
| + | :So, the area of the 10 things, which is DZ, is thirty. | ||
| + | :<math>\scriptstyle{\color{blue}{DZ=10x=30}}</math> | ||
| + | |style="text-align:right;"|א"כ שטח הי' דברים שהוא ד"ז יהיו שלשים | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|עוד רצוני להראותך התקון הנזכר באופן אחר | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|בהיות כי הדבר שהוא צלע הצינסו יהיה פעמים רבות יותר ממחצית הדברים שרש הנשאר בהוצאת הדברים המספרים או שטחו משטח מחצית כמות הדברים תכפול בעצמו בזה האופן אשר נצייר | ||
| + | |- | ||
| + | | | ||
| + | :[[File:Dardi 4.png|thumb|200px|left]] | ||
| + | |[[File:דארדי 4.png|thumb|200px|right]] | ||
| + | |- | ||
| + | | | ||
| + | :The side of the square is AB. | ||
| + | :<math>\scriptstyle{\color{blue}{AB=x}}</math> | ||
| + | |style="text-align:right;"|צלע הצינסו יהיה א"ב | ||
| + | |- | ||
| + | | | ||
| + | :BD, GD, GA are perpendicular and equal to each other. | ||
| + | :<math>\scriptstyle{\color{blue}{BD=GD=GA\quad BD\perp GD\perp GA}}</math> | ||
| + | |style="text-align:right;"|וב"ד וג"ד וג"א כל אחד מהם נצב הזויות ושוים זה לזה | ||
| + | |- | ||
| + | | | ||
| + | :The surface of the square is GB. | ||
| + | :<math>\scriptstyle{\color{blue}{GB=x^2}}</math> | ||
| + | |style="text-align:right;"|שטח זה הצינסו הוא ג"ב | ||
| + | |- | ||
| + | | | ||
| + | :We add 21, which is surface BL, to the square | ||
| + | :<math>\scriptstyle{\color{blue}{BL=21}}</math> | ||
| + | |style="text-align:right;"|ונוסיף על זה הצינסו כ"א והוא שטח ב"ל | ||
| + | |- | ||
| + | | | ||
| + | :The width of this surface is line BW, and its length is as the thing, or say as the side of the square, that is equal to WL. | ||
| + | :<math>\scriptstyle{\color{blue}{WL=x=\sqrt{x^2}}}</math> | ||
| + | |style="text-align:right;"|וזה השטח מרחבו קו ב"ו ומאורך כמו הדבר או אמור כצלע הצינסו אשר הוא שוה לו"ל | ||
| + | |- | ||
| + | | | ||
| + | :We receive that these two surfaces, which are GB and DW, are equal to ten things. | ||
| + | :<math>\scriptstyle{\color{blue}{GB+DW=10x}}</math> | ||
| + | |style="text-align:right;"|ויהיו לנו שני אלו השטחים שהם ג"ב וד"ו שוים לעשרה דברים | ||
| + | |- | ||
| + | | | ||
| + | :The length of these 10 things is from A to W. | ||
| + | |style="text-align:right;"|אשר אלו הי' דברים יש להם מאורך מא' עד ו‫' | ||
| + | |- | ||
| + | | | ||
| + | :We cut this length into two equal parts at point H. | ||
| + | |style="text-align:right;"|וזה האורך אנו {{#annot:to halve a line at point|820,1369|yo7t}}נחצה לשני חלקים שוים בנקודת{{#annotend:yo7t}} ה‫' | ||
| + | |- | ||
| + | | | ||
| + | :So, we have AH and HW that are five. | ||
| + | :<math>\scriptstyle{\color{blue}{AH=HW=5}}</math> | ||
| + | |style="text-align:right;"|א"כ יש לנו א"ה וכן ה"ו יהיו חמשה | ||
| + | |- | ||
| + | | | ||
| + | :We draw a straight line HE from H to E, which is five. | ||
| + | :<math>\scriptstyle{\color{blue}{HE=5}}</math> | ||
| + | |style="text-align:right;"|ואורך ה"ו {{#annot:to draw a line|819,1232|yF7x}}נוציא קו{{#annotend:yF7x}} אחד ישר מה' אל ע' ויהיה ה"ע חמשה | ||
| + | |- | ||
| + | | | ||
| + | :We draw another straight line [EC] from E to C parallel to line WH. | ||
| + | :<math>\scriptstyle{\color{blue}{\left[EC\right]\parallel WH}}</math> | ||
| + | |style="text-align:right;"|ו{{#annot:to draw a line|819,1232|Z0HS}}נוציא קו אחר ישר{{#annotend:Z0HS}} מע' אל ח' {{#annot:term|825,1821|dReT}}נכחי ל{{#annotend:dReT}}קו ו"ה | ||
| + | |- | ||
| + | | | ||
| + | :We get an equilateral right-angled surface [= EW], HW, WC, CE, EH. | ||
| + | |style="text-align:right;"|ויהיה לנו שטח אחד שוה הצלעות ונצב הזויות עליו ה"ו ו"ח ח"ע ע"ה | ||
| + | |- | ||
| + | | | ||
| + | :Each of these sides is five. | ||
| + | :<math>\scriptstyle{\color{blue}{HW=WC=CE=EH=5}}</math> | ||
| + | |style="text-align:right;"|ויהיה כל אחד מאלו הצלעות חמשה | ||
| + | |- | ||
| + | | | ||
| + | :The area of this square is 25. | ||
| + | :<math>\scriptstyle{\color{blue}{\left[EW\right]=25}}</math> | ||
| + | |style="text-align:right;"|ושטח זה המרובע יהיה כ"ה | ||
| + | |- | ||
| + | | | ||
| + | :Hence, surface EW is greater than DW by 4. | ||
| + | :<math>\scriptstyle{\color{blue}{EW=4+DW}}</math> | ||
| + | |style="text-align:right;"|א"כ שטח ע"ו יהיה ד' יותר מד"ו | ||
| + | |- | ||
| + | | | ||
| + | :Likewise, we get surface EB that is greater than DC by 4. | ||
| + | :<math>\scriptstyle{\color{blue}{EB=4+DC}}</math> | ||
| + | |style="text-align:right;"|וכדומה לזה יהיה לנו שטח ע"ב היותו יותר ד' מד"ח | ||
| + | |- | ||
| + | | | ||
| + | :So, we have one straight line SP equal to EK. | ||
| + | :<math>\scriptstyle{\color{blue}{SP=EK}}</math> | ||
| + | |style="text-align:right;"|א"כ יהיה לנו קו אחד ישר מס"פ שוה לע"כ | ||
| + | |- | ||
| + | | | ||
| + | :Now, we have lines SN and PD that are equal to EC. | ||
| + | :<math>\scriptstyle{\color{blue}{SN=PD=EC}}</math> | ||
| + | |style="text-align:right;"|ועתה יהיה לנו קו ס"נ ופ"ד שוה לע"ח | ||
| + | |- | ||
| + | | | ||
| + | :Since LW exceeds CW as DB exceeds DP. | ||
| + | :<math>\scriptstyle{\color{blue}{LW-CW=DB-DP}}</math> | ||
| + | |style="text-align:right;"|מפני כי כל כך {{#annot:term|420,2035|x2qf}}יעדיף{{#annotend:x2qf}} ל"ו על ח"ו כמו שיעדיף ד"ב אל ד"פ | ||
| + | |- | ||
| + | | | ||
| + | :So, AB exceeds AH as DB exceeds DP, or LW exceeds WC. | ||
| + | :<math>\scriptstyle{\color{blue}{AB-AH=DB-DP=LW-WC}}</math> | ||
| + | |style="text-align:right;"|וככה יעדיף א"ב על א"ה כמו ד"ב על ד"פ או ל"ו אל ו"ח | ||
| + | |- | ||
| + | | | ||
| + | :BS□ = [?] | ||
| + | |style="text-align:right;"|א"כ שטח ב"ס יהיה שוה לשטח | ||
| + | |- | ||
| + | | | ||
| + | :EP is equal to surface DC. | ||
| + | :<math>\scriptstyle{\color{blue}{EP=DC}}</math> | ||
| + | |style="text-align:right;"|ע"פ הוא שוה לשטח ד"ח | ||
| + | |- | ||
| + | | | ||
| + | :Surface DW is 21. | ||
| + | :<math>\scriptstyle{\color{blue}{DW=21}}</math> | ||
| + | |style="text-align:right;"|ושטח ד"ו הוא כ"א | ||
| + | |- | ||
| + | | | ||
| + | :So, these two surfaces, which are EP plus KW, are 21. | ||
| + | :<math>\scriptstyle{\color{blue}{EP+KW=21}}</math> | ||
| + | |style="text-align:right;"|א"כ אלו שני השטחים שהם ע"פ כ"ו יהיו כ"א | ||
| + | |- | ||
| + | | | ||
| + | :Surface SB is 4 and its side is a root of 4. | ||
| + | :<math>\scriptstyle{\color{blue}{SB=4}}</math> | ||
| + | |style="text-align:right;"|ושטח ס"ב הוא ד' וצלעו יהיה שרש ד‫' | ||
| + | |- | ||
| + | | | ||
| + | :AH is five. since HW is five, which is the length of half the number of the things. | ||
| + | :<math>\scriptstyle{\color{blue}{AH=HW=\frac{1}{2}\sdot10=5}}</math> | ||
| + | |style="text-align:right;"|וא"ה הוא חמשה<br> | ||
| + | מפני כי ה"ו הוא חמשה שהוא אורך מחצית כמות הדברים | ||
| + | |- | ||
| + | | | ||
| + | :Therefore, AB is five plus a root of 4 and this is equal to the thing. | ||
| + | :<math>\scriptstyle{\color{blue}{x=AB=5+\sqrt{4}}}</math> | ||
| + | |style="text-align:right;"|אם כן א"ב הוא חמשה ושרש ד' וככה יהיה שוה הדבר | ||
| + | |- | ||
| + | | | ||
| + | :The square is its product by itself. | ||
| + | :<math>\scriptstyle{\color{blue}{x^2=\left(5+\sqrt{4}\right)^2}}</math> | ||
| + | |style="text-align:right;"|והצינסו הוא הכאתו בעצמו | ||
| + | |- | ||
| + | | | ||
| + | :When its total is converted to an expressible number, the thing is 7, because a root of 4 is 2. | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{4}=2\longrightarrow x=7}}</math> | ||
| + | |style="text-align:right;"|וכאשר הושב סכומו במספר מדובר יהיה הדבר ז‫'<br> | ||
| + | מפני כי שרש ד' הוא ב‫' | ||
| + | |- | ||
| + | | | ||
| + | :The square of 7 is 49, which is surface GB. | ||
| + | :<math>\scriptstyle{\color{blue}{x^2=GB=7^2=49}}</math> | ||
| + | |style="text-align:right;"|והצינסו מז' הוא מ"ט שטחו אשר עליו ג"ב | ||
| + | |- | ||
| + | |We illustrate the third compound chapter this way: | ||
| + | |style="text-align:right;"|הפרק השלישי המורכב אנחנו נראהו בצורה בזה האופן | ||
| + | |- | ||
| + | | | ||
| + | *We suppose we have one square that is equal to 3 things, or its 3 roots, plus 4 drami. | ||
| + | :<math>\scriptstyle x^2=3x+4</math> | ||
| + | |style="text-align:right;"|נניח שאנחנו יש לנו צינסו אחד שוה לג' דברים או ג' שרשיו וד' דרמי יותר | ||
| + | |- | ||
| + | | | ||
| + | :[[File:Dardi 5.png|thumb|170px|left]] | ||
| + | |[[File:דארדי 5.png|thumb|170px|right]] | ||
| + | |- | ||
| + | | | ||
| + | :We suppose the square is a surface whose sides are AB, BD, DZ, ZA and its surface is AD. | ||
| + | :<math>\scriptstyle{\color{blue}{AD=x^2}}</math> | ||
| + | |style="text-align:right;"|אנו נניח שהצינסו יהיה שטח על צלעיו א"ב ב"ד ד"ז ז"א ושטחו יהיה א"ד | ||
| + | |- | ||
| + | | | ||
| + | :We assume that its 3 roots, which together with the 4 drami are equal to the whole square, are from Z to H. | ||
| + | |style="text-align:right;"|ונעשה שמז' עד ה' יהיו ג' שרשיו אשר עם ד' דרמי יהיה שוה לכל הסינסו | ||
| + | |- | ||
| + | | | ||
| + | :We draw line [BP] parallel to AH. | ||
| + | :<math>\scriptstyle{\color{blue}{\left[BP\right]\parallel AH}}</math> | ||
| + | |style="text-align:right;"|ונוציא קו אחד נכחי לא"ה | ||
| + | |- | ||
| + | | | ||
| + | :So, surface HD is the 3 things. | ||
| + | :<math>\scriptstyle{\color{blue}{HD=3x}}</math> | ||
| + | |style="text-align:right;"|א"כ שטח ה"ד יהיה הג' דברים | ||
| + | |- | ||
| + | | | ||
| + | :Surface HB is 4 drami, because when we add 4 to the 3 things, it equals the whole square. | ||
| + | :<math>\scriptstyle{\color{blue}{\left[HB+HD\right]=4+3x=x^2=\left[AD\right]\longrightarrow HB=4}}</math> | ||
| + | |style="text-align:right;"|ושטח ה"ב יהיה ד' דרמי<br> | ||
| + | מפני כי כשנוסיף ד' על ג' דברים הוא שוה לכל הצינסו | ||
| + | |- | ||
| + | | | ||
| + | :Cut line HZ, whose length is 3, into two equal parts, so we get that from Z to C, or from H to C it is one and a half. | ||
| + | :<math>\scriptstyle{\color{blue}{ZC=HC=\frac{1}{2}HZ=\frac{1}{2}\sdot3=1+\frac{1}{2}}}</math> | ||
| + | |style="text-align:right;"|אח"כ תחלק קו ה"ז לשני חלקים שוים אשר זה הקו ארכו שלשה דברים ויש לנו מז' עד ח' או מה' עד ח' אחד וחצי | ||
| + | |- | ||
| + | | | ||
| + | :Then, draw parallel lines, one from H to K, and one from C to T, the length of each is equal to HC, which is one and a half. | ||
| + | :<math>\scriptstyle{\color{blue}{\left[HK\right]\parallel\left[CT\right]\quad HK=CT=HC=1+\frac{1}{2}}}</math> | ||
| + | |style="text-align:right;"|אח"כ {{#annot:to draw a line|819,1232|cMHc}}תוציא קו אחד מ{{#annotend:cMHc}}ה' אל כ' ואחד מח' אל ט' {{#annot:term|825,1821|lKms}}נכחיים{{#annotend:lKms}} אשר יהיה אורך כל אחד שוה לה"ח שהוא אחד וחצי | ||
| + | |- | ||
| + | | | ||
| + | :So, when we draw line CT, we get an equilateral quadrilateral [= TH]. | ||
| + | |style="text-align:right;"|א"כ כשנוציא קו ח"ט יש לנו {{#annot:term|305,1863|94Yd}}מרובע שוה הצלעות{{#annotend:94Yd}} | ||
| + | |- | ||
| + | | | ||
| + | :Since line KH is equal to HC; and HC is equal to CT or HK; and each of these sides is one and a half. | ||
| + | :<math>\scriptstyle{\color{blue}{KH=HC=CT=HK=1+\frac{1}{2}}}</math> | ||
| + | |style="text-align:right;"|מפני כי קו כ"ה שוה לה"ח וה"ח שוה לח"ט או לה"כ וכל אחד מאלו הצלעות יהיה אחד וחצי | ||
| + | |- | ||
| + | | | ||
| + | :Therefore, its area is 2 and a quarter and it is surface TH. | ||
| + | :<math>\scriptstyle{\color{blue}{TH=2+\frac{1}{4}}}</math> | ||
| + | |style="text-align:right;"|א"כ שטחו יהיה ב' ורביע והוא שטח ט"ה | ||
| + | |- | ||
| + | | | ||
| + | :I also want to add to line CT a line up to point L that would be equal to the AH, so that CL is equal to CA. | ||
| + | :<math>\scriptstyle{\color{blue}{CL=CA}}</math> | ||
| + | |style="text-align:right;"|עוד רציתי להוסיף על קו ח"ט קו אחד עד נקודת ל' שיהיה שוה לא"ה ויהיה ח"ל שוה לח"א | ||
| + | |- | ||
| + | | | ||
| + | :Now, draw line [LM] from L to M parallel to line CA. | ||
| + | :<math>\scriptstyle{\color{blue}{\left[LM\right]\parallel CA}}</math> | ||
| + | |style="text-align:right;"|ועתה תוציא קו אחד מל' בנקודת מ' נכחי לקו ח"א | ||
| + | |- | ||
| + | | | ||
| + | :It is equal to line CA, which is equal to CL and also to AM. | ||
| + | :<math>\scriptstyle{\color{blue}{\left[LM\right]=CA=CL=AM}}</math> | ||
| + | |style="text-align:right;"|ויהיה שוה לקו ח"א והוא שוה לח"ל וכן לא"מ | ||
| + | |- | ||
| + | | | ||
| + | :We receive an equilateral quadrilateral, which is surface CM. | ||
| + | |style="text-align:right;"|ויהיה לנו מרובע שוה הצלעות והוא שטח ח"מ | ||
| + | |- | ||
| + | | | ||
| + | :Since AM is equal to AC, MB is equal to CZ | ||
| + | :<math>\scriptstyle{\color{blue}{AM=AC\longrightarrow MB=CZ}}</math> | ||
| + | |style="text-align:right;"|ומפני שא"מ הוא שוה לא"ח יהיה מ"ב שוה לח"ז | ||
| + | |- | ||
| + | | | ||
| + | :CZ is equal to HC and HC is equal to CT, therefore MB is equal to CT. | ||
| + | :<math>\scriptstyle{\color{blue}{CZ=HC;\; HC=CT\longrightarrow MB=CT}}</math> | ||
| + | |style="text-align:right;"|וח"ז הוא שוה לה"ח וה"ח שוה לח"ט אם כן מ"ב הוא שוה לכ"ט | ||
| + | |- | ||
| + | | | ||
| + | :MN is equal to AH and AH is equal to TL, so MN is equal to TL | ||
| + | :<math>\scriptstyle{\color{blue}{MN=AH;\; AH=TL\longrightarrow MN=TL}}</math> | ||
| + | |style="text-align:right;"|והיה לנו כי מ"נ הוא שוה לא"ה וא"ה הוא שוה לט"ל<br> | ||
| + | א"כ מ"נ הוא שוה לט"ל | ||
| + | |- | ||
| + | | | ||
| + | :Surface KL is equal to surface NB. | ||
| + | :<math>\scriptstyle{\color{blue}{KL=NB}}</math> | ||
| + | |style="text-align:right;"|ושטח כ"ל יהיה שוה לשטח נ"ב | ||
| + | |- | ||
| + | | | ||
| + | :Therefore, surface AN plus surface KL are four, because these two surfaces are equal to surface HB, which is four. | ||
| + | :<math>\scriptstyle{\color{blue}{AN+KL=HB=4}}</math> | ||
| + | |style="text-align:right;"|א"כ שטח א"נ ושטח כ"ל יהיו ארבעה<br> | ||
| + | כי שני אלו השטחים א"נ וכ"ל יהיו שוים לשטח ה"ב שהוא ארבעה | ||
| + | |- | ||
| + | | | ||
| + | :So, surface CM is six and a quarter and its side is its root, because it is a square surface. | ||
| + | :<math>\scriptstyle{\color{blue}{CM=6+\frac{1}{4}}}</math> | ||
| + | |style="text-align:right;"|אם כן שטח ח"מ יהיה ששה ורביע וצלעו יהיה שרשו<br> | ||
| + | מפני כי הוא {{#annot:term|305,1863|0caF}}שטח אחד שוה הצלעות{{#annotend:0caF}} | ||
| + | |- | ||
| + | | | ||
| + | :Its side AC then is a root of six and a quarter, which is 2 and a [half]. | ||
| + | :<math>\scriptstyle{\color{blue}{AC=\sqrt{6+\frac{1}{4}}=2+\frac{1}{{\color{red}{2}}}}}</math> | ||
| + | |style="text-align:right;"|אם כן צלעו א"ח יהיה שרש ששה ורביע שהוא ב' ורביע | ||
| + | |- | ||
| + | | | ||
| + | :CZ is one and a half. | ||
| + | :<math>\scriptstyle{\color{blue}{CZ=1+\frac{1}{2}}}</math> | ||
| + | |style="text-align:right;"|ומח"ז הוא אחד וחצי | ||
| + | |- | ||
| + | | | ||
| + | :Therefore, the whole line AZ, which is a side of the square, or the thing, is four, or a root of 6 and a quarter plus 1 and a half, as you have seen in the figure drawn above. | ||
| + | :<math>\scriptstyle{\color{blue}{AZ=x=\sqrt{6+\frac{1}{4}}+\left(1+\frac{1}{2}\right)=4}}</math> | ||
| + | |style="text-align:right;"|שיהיה אם כן כל הקו מא"ז שהוא צלע הצינסו או הדבר יהיה ארבעה במספר או שרש ו' ורביע ויותר א' וחצי כמו שהראית בצורה הרשומה למעלה | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|בכאן נראה האופן והדרך להשיב השאלות אל ההשואות כאשר תוכל לראות | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== <span style=color:Green>Operations with Algebraic Expressions</span> ==== | ||
| + | |||
| + | | | ||
| + | |- | ||
| + | |Before giving any question, the way and the method are stated, which should be held in order to find the explanation how the questions are restored to the said chapters as well as other chapters that will be given from here on. | ||
| + | |style="text-align:right;"|קודם שאניח שום שאלה רצוני להגיד הדרך והאופן הצריך להחזיק בו כדי למצוא באור השאלות כיצד יושבו להשואת הפרקים האמורים וג"כ לפרקי' אחרי' אשר יונחו מכאן ולהבא | ||
| + | |- | ||
| + | |Knowing that what is unknown of the given question should always be defined as a thing or a çenso. | ||
| + | |style="text-align:right;"|ביודעך לעולם כי אשר אינך יודע מן השאלה המונחת הנך צריך להניח שיהיה דבר או צינסו | ||
| + | |- | ||
| + | |In any case, it should first be defined as a thing. | ||
| + | |style="text-align:right;"|ומכל מקום תניח ראשונה שיהיה דבר | ||
| + | |- | ||
| + | |Yet, many times it would be easier in the question that the unknown term will be çenso. | ||
| + | |style="text-align:right;"|ופעמים רבות בשאלה יגיעך נקל יותר שהחלק הנעלם יהיה צינסו | ||
| + | |- | ||
| + | |Since, defining the thing instead of a çenso changes the chapter or the equation. | ||
| + | |style="text-align:right;"|בהיות כי מהנחת הדבר במקום צינסו יגיע חילוף הפרק או ההשואה | ||
| + | |- | ||
| + | |As seen above: thing = the side of the çenso, or √çenso | ||
| + | |style="text-align:right;"|וכמו שהראינו לפנים הדבר הוא צלע הצינסו או שרש הצינסו | ||
| + | |- | ||
| + | |Therefore: | ||
| + | *thing × thing = çenso <math>\scriptstyle x\times x=x^2</math> | ||
| + | |style="text-align:right;"|ולכן בהכותנו או {{#annot:term|185,1230|7joL}}בכפלנו{{#annotend:7joL}} הדבר בעצמו עושה הצינסו | ||
| + | |- | ||
| + | | | ||
| + | *thing × çenso = cube <math>\scriptstyle x\times x^2=x^3</math> | ||
| + | |style="text-align:right;"|והדבר כשיוכה בצינסו עושה מעוקב | ||
| + | |- | ||
| + | |According to these three names, the number is understood in three ways: | ||
| + | |style="text-align:right;"|ובאלו הג' שמות יובן המספר בג' אופנים | ||
| + | |- | ||
| + | | | ||
| + | *the length of the line | ||
| + | |style="text-align:right;"|או כפי האורך הקו | ||
| + | |- | ||
| + | | | ||
| + | *the area of the surface | ||
| + | |style="text-align:right;"|או כפי {{#annot:term|816,1488|n6dR}}רחב השטח{{#annotend:n6dR}} | ||
| + | |- | ||
| + | | | ||
| + | *the volume which is a corporeal quantity | ||
| + | |style="text-align:right;"|או כפי העובי שהוא כמות גשמי | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|בהיות כי הראינו לך הדבר והצינסו בצורה שעבר | ||
| + | |- | ||
| + | | | ||
| + | *thing = length of the side of the çenso | ||
| + | |style="text-align:right;"|רצוני שהדבר הוא אורך צלע הצינסו | ||
| + | |- | ||
| + | | | ||
| + | *çenso = area of a square surface | ||
| + | |style="text-align:right;"|והצינסו הוא {{#annot:term|305,2560|va50}}שטח שוה הצלעות נצב הזויות{{#annotend:va50}} | ||
| + | |- | ||
| + | | | ||
| + | *cube: its width, length and height are equal by its nature | ||
| + | |style="text-align:right;"|רצוני לאמר לך מן המעוקב טבעו כי הוא רחב ואורך וגובה שוים יחד | ||
| + | |- | ||
| + | | | ||
| + | ::as the shape of the cube which is equal by length, width and height | ||
| + | |style="text-align:right;"|כמו צורת הקוביא שהוא שוה בגובה וברחב ובארך | ||
| + | |- | ||
| + | | | ||
| + | :::its facets are equal surfaces | ||
| + | |style="text-align:right;"|וצדדיו יהיו משטחים שוים | ||
| + | |- | ||
| + | | | ||
| + | :::its sides have equal length | ||
| + | |style="text-align:right;"|וצלעותיו מאורך שוה | ||
| + | |- | ||
| + | | | ||
| + | :::its angels are equal to each other | ||
| + | |style="text-align:right;"|וכמו כן זויותיו יהיה שוים זה אל זה | ||
| + | |- | ||
| + | | | ||
| + | :each side of the cube = thing | ||
| + | |style="text-align:right;"|א"כ כל צלע מהמעוקב הוא הדבר | ||
| + | |- | ||
| + | | | ||
| + | :each of its facets = çenso | ||
| + | |style="text-align:right;"|וכל אחד מצדדיו יהיה הצינסו שלו | ||
| + | |- | ||
| + | | | ||
| + | :its principle = cube | ||
| + | |style="text-align:right;"|וראשיותיו הוא המעוקב | ||
| + | |- | ||
| + | | | ||
| + | *thing × thing = çenso <math>\scriptstyle x\times x=x^2</math> | ||
| + | |style="text-align:right;"|א"כ בהכפל דבר בדבר עושה צינסו | ||
| + | |- | ||
| + | | | ||
| + | *thing × çenso = cube <math>\scriptstyle x\times x^2=x^3</math> | ||
| + | |style="text-align:right;"|ובהכות דבר בצינסו עושה מעוקב | ||
| + | |- | ||
| + | | | ||
| + | *thing × cube = çenso of çenso <math>\scriptstyle x\times x^3=x^4</math> | ||
| + | |style="text-align:right;"|ודבר במעוקב עושה צינסו מצינסו | ||
| + | |- | ||
| + | |The çenso of çenso can be understood from two aspects: | ||
| + | |style="text-align:right;"|וזה הצינסו מצינסו אפשר להבינו בשני פנים | ||
| + | |- | ||
| + | | | ||
| + | :*çenso × çenso = çenso of çenso <math>\scriptstyle x^2\times x^2=x^4</math> | ||
| + | |style="text-align:right;"|בעבור כי צינסו מוכה בצינסו עושה צינסו מצינסו | ||
| + | |- | ||
| + | | | ||
| + | :*thing × [cube] = çenso of çenso <math>\scriptstyle x\times{\color{red}{x^3}}=x^4</math> | ||
| + | |style="text-align:right;"|ודבר מוכה בצינסו עושה צינסו מצינסו | ||
| + | |- | ||
| + | | | ||
| + | *when numbers are multiplied by a certain amount of things it yields as much things as the amount of the things multiplied by the number <math>\scriptstyle a\times bx=\left(a\sdot b\right)x</math> | ||
| + | |style="text-align:right;"|וכשיוכו מספרים בכמות מה מדברים עושה כל כך דברים כמו שעולה כמות הדברי' מוכה במספר | ||
| + | |- | ||
| + | | | ||
| + | *the product of a certain amount of çenso by a number yields as much çenso as the product of [the amount of the çenso] by the number multiplied <math>\scriptstyle ax^2\times b=\left(a\sdot b\right)x^2</math> | ||
| + | |style="text-align:right;"|ובהכות כמות מה מצינסי במספר עושה כל כך צינסו כמו שעולה ההכאה ההיא במספר המוכה | ||
| + | |- | ||
| + | | | ||
| + | *the product of a certain amount of cubes by a number yields as much cubes as the product of the said amount [of cubes] by the number <math>\scriptstyle ax^3\times b=\left(a\sdot b\right)x^3</math> | ||
| + | |style="text-align:right;"|ובהכותנו כמות מה מ{{#annot:term|688,1828|ag26}}מעוקבים{{#annotend:ag26}} במספר עושה כל כך מעוקבים כמו שעולה הכמות האמור במספר | ||
| + | |- | ||
| + | | | ||
| + | *<math>\scriptstyle ax^4\times b=\left(a\sdot b\right)x^4</math> | ||
| + | |style="text-align:right;"|ובהכפל כמות מה מ{{#annot:term|689|UeHi}}צינסי דצינסי{{#annotend:UeHi}} במספר עושה כל כך {{#annot:term|689|rRuk}}צינסי מצינסי{{#annotend:rRuk}} כמו שעולה כמות הצינסי מצינסי כפולים במספר | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ועם אלו הד' שמות אשר בארנו שהם דבר וצינסו ומעוקב וצינסו מצינסו יתפשטו הפרקי' אשר יכתבו כפי מה שתראה מכאן ולהבא | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ודע כי אם יזדמן לך לכפול מספר ודבר במספר ודבר או מספר וצינסו במספר וצינסו או מספר ודבר במספר פחות דבר או מספר וצינסו במספר פחות צינסו או בכל אופן אחר שיזדמן כפי שנויי ההכפלות שאפשר שיבאו תהיה ממשיך ההכפלה באופן אשר הראית למעלה בכפילת השרשים בהביטך לעולם להמשיך הטבע או ה{{#annot:term|1058|GY0W}}עצמויות{{#annotend:GY0W}} העושות הכמויות הנקובי' בשם בכפול האחד באחר כמו שכבר הראית קודם וכמו שנראך עתה בכמה המשלים | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|נניח שרצית לכפול דבר במספר | ||
| + | או דראמא בהיות כי האחדות אשר במספר יקראוהו דראמ‫' | ||
| + | |- | ||
| + | | | ||
| + | *<math>\scriptstyle{\color{blue}{3x\times4=12}}</math> | ||
| + | |style="text-align:right;"|לכן בכפול ג' דברים בד' דראמי יעלה י"ב דברי‫' | ||
| + | |- | ||
| + | | | ||
| + | *<math>\scriptstyle{\color{blue}{4x^2\times3=12x^2}}</math> | ||
| + | |style="text-align:right;"|וד' צינסו בג' דראמ' יעלה י"ב צינסו | ||
| + | |- | ||
| + | | | ||
| + | *<math>\scriptstyle{\color{blue}{2x^3\times5=10x^3}}</math> | ||
| + | |style="text-align:right;"|וב' מעוקבי' בה' דראמ' יעלה י' מעוקבי‫' | ||
| + | |- | ||
| + | | | ||
| + | *<math>\scriptstyle{\color{blue}{5x^4\times3=15x^4}}</math> | ||
| + | |style="text-align:right;"|וה' צינסי מצינסי בג' דראמ' יעלה ט"ו צינסו מצינסו | ||
| + | |- | ||
| + | |Multiplication of a Number and a Thing by a Number and a Thing | ||
| + | |style="text-align:right;"|ואם רצית לכפול מספר ודבר במספר ודבר | ||
| + | |- | ||
| + | | | ||
| + | *<math>\scriptstyle\left(3+2x\right)\times\left(3+2x\right)</math> | ||
| + | |style="text-align:right;"|נניח שרצית לכפול ג' וב' דברי' בג' וב' דברים | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|הנך צריך לרדוף הכלל מהכפל בשרשים כפי מה שהראית למעלה | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|הנך צריך לכפול המספרים זה על זה | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{3\sdot3=9}}</math> | ||
| + | |style="text-align:right;"|שהוא ג' בג' ועולה ט' ושמור | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|אח"כ תכה המספרי' בשתי וערב עם הדברים | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{3\sdot2x=6x}}</math> | ||
| + | |style="text-align:right;"|שהוא ג' בב' דברים ועולה ו' דברים | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{3\sdot2x=6x}}</math> | ||
| + | |style="text-align:right;"|ואח"כ הג' האחר בב' דברים האחרים ועולה ו' דברים | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{6x+6x=12x}}</math> | ||
| + | |style="text-align:right;"|ו{{#annot:term|178,1165|3DfE}}חברם יחד{{#annotend:3DfE}} ויהיה לך י"ב דברים ושמור | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|אח"כ תכפול הדברים זה בזה | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{2x\sdot2x=4x^2}}</math> | ||
| + | |style="text-align:right;"|דהיינו ב' דברי' בב' דברים ועולה ד' צינסו | ||
| + | |- | ||
| + | | | ||
| + | :<math>\scriptstyle{\color{blue}{\left(3+2x\right)\times\left(3+2x\right)=9+12x+4x^2}}</math> | ||
| + | |style="text-align:right;"|וחבר הכל יחד ויהיה לך סכומם ט' דראמ' וי"ב דברים וד' צינסי | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ובזה האופן תרדוף בשאר ה{{#annot:term|156,1229|w4ij}}הכפלות{{#annotend:w4ij}} | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|בכאן יראה האופן מהחלוק והסקיזארי מאלו המספרי' שהם דבר וצינסו ומעוקב וצינסו מצינסו כאשר תראה תחת זה | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|עתה רצוני להראות לך באי זה אופן יהיה החלוק והבצוע מאלו השמות שהם דבר וצינסו ומעוקב וצינסו מצינסו | ||
| + | |- | ||
| + | | | ||
| + | *thing ÷ number or drama = thing | ||
| + | |style="text-align:right;"|בהיות כי לחלוק או {{#annot:term|784,2520|OTlz}}לבצע{{#annotend:OTlz}} הדבר במספר או בדראמ' יגיע דבר | ||
| + | |- | ||
| + | | | ||
| + | ::number × thing = thing | ||
| + | |style="text-align:right;"|מפני כי בכפול מספר על דבר עושה דבר | ||
| + | |- | ||
| + | | | ||
| + | *çenso ÷ number = çenso | ||
| + | |style="text-align:right;"|ובחלק צינסו על מספר יגיע צינסו | ||
| + | |- | ||
| + | | | ||
| + | ::çenso × number = çenso | ||
| + | |style="text-align:right;"|מפני כי הצינסו מוכה במספר עושה צינסו | ||
| + | |- | ||
| + | | | ||
| + | *cube ÷ number or drama = cube | ||
| + | |style="text-align:right;"|ובחלק מעוקב על מספר או על דראמ' יהיה המגיע מעוקב | ||
| + | |- | ||
| + | | | ||
| + | ::cube × number = cube | ||
| + | |style="text-align:right;"|מפני כי המעוקב כש{{#annot:term|358,2025|QSst}}הוכה ב{{#annotend:QSst}}מספר עושה מעוקב | ||
| + | |- | ||
| + | | | ||
| + | *çenso of çenso ÷ number or drama = çenso of çenso | ||
| + | |style="text-align:right;"|ובחלוק או לבצע צינסו מצינסו על מספר או דראמ' יגיע צינסו מצינסו | ||
| + | |- | ||
| + | | | ||
| + | ::çenso of çenso × number = çenso of çenso | ||
| + | |style="text-align:right;"|מפני כי בכפול צינסו דצינסו על מספר עושה צינסו מצינסו | ||
| + | |- | ||
| + | | | ||
| + | *thing ÷ thing = number or drama | ||
| + | |style="text-align:right;"|ובחלוק דבר על דבר יגיע מספר או דראמ‫' | ||
| + | |- | ||
| + | | | ||
| + | *çenso ÷ çenso = drama | ||
| + | |style="text-align:right;"|ובחלוק צינסו דצינסו יגיע דראמ‫' | ||
| + | |- | ||
| + | | | ||
| + | *cube ÷ cube = number or drama | ||
| + | |style="text-align:right;"|ומעוקב על מעוקב יגיע מספר או דראמ‫' | ||
| + | |- | ||
| + | | | ||
| + | *çenso of çenso ÷ çenso of çenso = drama | ||
| + | |style="text-align:right;"|ובחלוק צינסו וצינסו דצינסו על צינסו דצינסו יגיע מספר או יהיה דראמ‫' | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|מפני כי בכפול כל אחד מאלו הכמויות על דראמ' או מספר עושה כמו כן אותו הכמות | ||
| + | |- | ||
| + | | | ||
| + | *<math>\scriptstyle x^2\div x=x</math> | ||
| + | |style="text-align:right;"|וכן לחלוק או לבצע צינסו בדבר יגיע דבר | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle x\times x=x^2</math> | ||
| + | |style="text-align:right;"|מפני כי הכאת דבר בדבר עושה צינסו | ||
| + | |- | ||
| + | | | ||
| + | *<math>\scriptstyle x^3\div x=x^2</math> | ||
| + | |style="text-align:right;"|ולחלוק מעוקב על דבר יגיע צינסו | ||
| + | |- | ||
| + | | | ||
| + | *<math>\scriptstyle x^3\div x^2=x</math> | ||
| + | |style="text-align:right;"|ולחלוק מעוקב על צינסו יגיע ממנו דבר | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle x\times x^2=x^2\times x= x^3</math> | ||
| + | |style="text-align:right;"|מפני כי דבר כשהוכה בצינסו או צינסו מוכה בדבר עושה מעוקב | ||
| + | |- | ||
| + | | | ||
| + | *<math>\scriptstyle x^4\div x=x^3</math> | ||
| + | |style="text-align:right;"|ולחלוק צינסו מצינסו על דבר יהיה המגיע מעוקב | ||
| + | |- | ||
| + | | | ||
| + | *<math>\scriptstyle x^4\div x^2=x^2</math> | ||
| + | |style="text-align:right;"|ולחלוק צינסו מצינסו על צינסו יגיע ממנו צינסו | ||
| + | |- | ||
| + | | | ||
| + | *<math>\scriptstyle x^4\div x^3=x</math> | ||
| + | |style="text-align:right;"|ולחלוק צינסו דצינסו על מעוקב יגיע ממנו דבר | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle x\times x^3=x^2\times x^2=x^3\times x=x^4</math> | ||
| + | |style="text-align:right;"|מפני כי בכפול דבר במעוקב או צינסו בצינסו או מעוקב בדבר עושה צינסו מצינסו | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|אם כן כל כמות כשהוכפל באחר הנה העולה ממנו כאשר {{#annot:term|784,1226|ci6L}}נחלק על{{#annotend:ci6L}} אחד מכמויות ההם עושה הכמות האחר | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|והנה לך למשל במספר | ||
| + | |- | ||
| + | | | ||
| + | :*<math>\scriptstyle{\color{blue}{4\times3=12}}</math> | ||
| + | |style="text-align:right;"|כשהוכפל ד' אחדים על ג' אחדים עושה י"ב אחדים | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{12\div4=3}}</math> | ||
| + | |style="text-align:right;"|ולחלוק י"ב על הראשון שהוא ד' יגיע ממנו הכמות השני שהוא ג‫' | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{12\div3=4}}</math> | ||
| + | |style="text-align:right;"|ובחלוק י"ב בכמות השני שהוא ג' יגיע ממנו הכמות הראשון שהוא ד‫' | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|דע כי בכאן בקרוב יגלה ההרגל מהו' פרקי' מאלגֵ'בְלֵי אמוגאבאלא כמו שתוכל לראות במשל | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== Chapter One ==== | ||
| + | |||
| + | |style="width:45%;text-align:right;"|<big>פרק ראשון</big> | ||
| + | |- | ||
| + | |When things are equal to numbers. | ||
| + | :<math>\scriptstyle bx=c</math> | ||
| + | |style="text-align:right;"|כאשר הדברים יהיו שוים אל המספרי‫' | ||
| + | |- | ||
| + | |The number should be divided by the things and the result is the thing. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{x=\frac{c}{b}}}</math> | ||
| + | |style="text-align:right;"|צריך לחלק המספר על הדברי‫'<br> | ||
| + | והעולה יהיה מספר וככה שוה הדבר | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:a+b=10, b²-a²=50|619|M4QJ}}Question: for example, divide ten into two parts, such that when each part is multiplied by itself, then the smaller product is subtracted from the greater, 10 remain. | ||
| + | :How much will each one of the parts be? | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle a+b=10\\\scriptstyle b^2-a^2=50\end{cases}</math> | ||
| + | |style="text-align:right;"|שאלה למשל עשה לי מעשרה שני חלקים באופן כי כשהוכה כל חלק בעצמו ויגרע קטון ההכאות מהגדולה ישאר חמשים<br> | ||
| + | א"כ כמה יהיה כל חלק מאלו החלקים{{#annotend:M4QJ}} | ||
| + | |- | ||
| + | | | ||
| + | :This is its rule according to al-Jīblī al-Mūqabāla: | ||
| + | |style="text-align:right;"|זהו כללו כפי אלג'בלי אמוג'בלה | ||
| + | |- | ||
| + | | | ||
| + | :Suppose one of its parts is a thing [<math>\scriptstyle{\color{blue}{a=x}}</math>], so the other remains ten minus a thing [<math>\scriptstyle{\color{blue}{b=10-x}}</math>]. | ||
| + | : | ||
| + | |style="text-align:right;"|נניח כי אחד מחלקיו יהיה דבר אחד והחלק האחר א"כ ישאר עשרה פחות דבר אחד | ||
| + | |- | ||
| + | | | ||
| + | :Multiply a thing by a thing; the result is one square. | ||
| + | :<math>\scriptstyle{\color{blue}{x\sdot x=x^2}}</math> | ||
| + | |style="text-align:right;"|עתה תכה דבר אחד בדבר אחד ועולה צינסו אחד | ||
| + | |- | ||
| + | | | ||
| + | :Then, multiply the other part, which is ten minus a thing, by itself; the result is 100 numbers or drami and one square minus 20 things. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(10-x\right)^2=100+x^2-20x}}</math> | ||
| + | |style="text-align:right;"|אח"כ תכה החלק האחר שהוא עשרה פחות דבר אחד על עצמו שעולה ק' מספרים או דראמי וצינסו אחד פחות כ' דברים | ||
| + | |- | ||
| + | | | ||
| + | :Now, subtract the product of the first [by itself], which is 1 square, from the product of the greater part by itself, which is 100 numbers and 1 square minus 20 things; 100 numbers minus 20 things remain, and this remainder is equal to 50 numbers according to the stated question. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(100+x^2-20x\right)-x^2=100-20x=50}}</math> | ||
| + | |style="text-align:right;"|עתה תוציא החלק האחד המוכה שהוא א' צינסו חוצה מזה הסך מהכאת החלק הגדול בעצמו שהוא ק' מספרים וא' צינסו פחות כ' דברים וישאר ק' מספרים פחות כ' דברים וזה הנשאר הוא שוה לנ' מספרים כפי השאלה הנזכרת | ||
| + | |- | ||
| + | | | ||
| + | :See that when the sides are equal, one of the sides is minus 20 things. | ||
| + | |style="text-align:right;"|עתה התבונן כי בהיות החלקי' שוים מאחד מהחלקי' יהיו כ' דברים פחות | ||
| + | |- | ||
| + | | | ||
| + | :So, when 20 things are given to each of the sides - as requires the rule of the six chapters that says: when something is subtracted from one side, the subtracted should always be added to it and [it] should be added to the other side as well - when we add 20 things to each of the sides, they are [still] equal. | ||
| + | |style="text-align:right;"|אם כן בהנתן כ' דברים לכל אחד מהחלקי' כאשר רוצה הכלל מהו' פרקי' האמורים האומ' כאשר יחסר מחלק מה אי זה דבר צריך להוסיף עליו לעולם מה שיחסר וכן צריך להוסיף מחלק האחד כמו לאחר א"כ בהוסיפנו כ' דברים לכל אחד מהחלקים ההם יהיו שוים | ||
| + | |- | ||
| + | | | ||
| + | :<span style=color:Green>'''Restoration''':</span> Therefore, add 20 things to 100 numbers minus 20 things; one of the sides becomes exactly 100 numbers. | ||
| + | :<math>\scriptstyle{\color{blue}{100-20x+20x=100}}</math> | ||
| + | |style="text-align:right;"|ולכן תוסיף כ' דברים על ק' מספרים פחות כ' דברים שיהיה אחד מהחלקים ויהיה ק' מספרים בדיוק | ||
| + | |- | ||
| + | | | ||
| + | :Add 20 things to the other side, which is 50. | ||
| + | :<math>\scriptstyle{\color{blue}{50+20x}}</math> | ||
| + | |style="text-align:right;"|ועל החלק האחר שהוא נ' תוסיף כ' דברים | ||
| + | |- | ||
| + | | | ||
| + | :Now, we have 100 numbers that are equal to 50 numbers and 20 things. | ||
| + | :<math>\scriptstyle{\color{blue}{100=50+20x}}</math> | ||
| + | |style="text-align:right;"|ועתה יהיה לנו כי ק' מספרים יהיו שוי' לנ' מספרי' וכ' דברי‫' | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{100-50}}</math> | ||
| + | |style="text-align:right;"|ועתה צריך להוציא המספר הקטן מהגדול שהוא נ' מק‫' | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{50=20x}}</math> | ||
| + | |style="text-align:right;"|וישאר מהחלק האחד נ' מספרי' ומן החלק האחר כ' דברי' לבד | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{100-50=50+20x-50}}</math> | ||
| + | |style="text-align:right;"|מפני כי בהיות החלקי' שוים הוצאת נ' מן החלק האחד כמו מהאחר | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{50=20x}}</math> | ||
| + | |style="text-align:right;"|וישאר א"כ נ' מספרי' שוים לכ' הדברי‫' | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{a=x=\frac{50}{20}=2+\frac{1}{2}}}</math> | ||
| + | |style="text-align:right;"|והם אנו צריכי' לחלק כפי הכלל האמור למעלה המספרי' על הדברים דהיינו נ' על כ' ו{{#annot:term|875,1566|c7MZ}}יבא{{#annotend:c7MZ}} ב' וחצי וככה שוה הדבר שהוא אחד מחלקי עשרה | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{b=10-x=10-\left(2+\frac{1}{2}\right)=7+\frac{1}{2}}}</math> | ||
| + | |style="text-align:right;"|והאחר ישאר עשרה פחות זה הדבר שהוא ב' וחצי וישאר ז' וחצי וכן הוא החלק האחר | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{a=2+\frac{1}{2}}}</math> | ||
| + | |style="text-align:right;"|א"כ יהיה לך כי אחד מחלקי עשרה הוא ב' וחצי | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{b=7+\frac{1}{2}}}</math> | ||
| + | |style="text-align:right;"|והחלק האחר יהיה ז' וחצי | ||
| + | |- | ||
| + | |Another example: | ||
| + | |style="text-align:right;"|עוד רצו' להניח חשבון אחר אל הפרק הזה הראשון | ||
| + | |- | ||
| + | | | ||
| + | *I wanted to buy 6 cubits of cloth of two types: one of the types is at 56 dinar for a cubit, and the other is at 67 dinar for a cubit. | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle a+b=6\\\scriptstyle 56a+67b=370\end{cases}</math> | ||
| + | |style="text-align:right;"|ואומ' כך כי רציתי לקנות ו' אמות בגד מב' מינים אחד מהחלקי' או מיני' מנ"ו דינרים האמה והאחר מס"ז דינרי' האמה | ||
| + | |- | ||
| + | | | ||
| + | ::of the one at 56 dinar: <math>\scriptstyle{\color{blue}{a=x}}</math> cubits | ||
| + | |style="text-align:right;"|תניח מהכלל האמור ואמור כן אני רוצה דבר אחד מאמות מנ"ו דינרי‫' | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{56a=56x}}</math> dinar | ||
| + | |style="text-align:right;"|שיהיה נ"ו דברים מדינרי‫' | ||
| + | |- | ||
| + | | | ||
| + | ::of the one at 67 dinar: <math>\scriptstyle{\color{blue}{b=6-x}}</math> cubits | ||
| + | |style="text-align:right;"|ואשאר לקחת ו' אמות פחות דבר אחד מס"ז דינרי' האמה | ||
| + | |- | ||
| + | | | ||
| + | ::the total price of the cloth = <math>\scriptstyle{\color{blue}{370}}</math> dinar | ||
| + | |style="text-align:right;"|וכל זה הבגד עלה לי ש"ע דינרי‫' | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{56x}}</math> | ||
| + | |style="text-align:right;"|אבל מפני כי אני מניח שדבר אחד מאמות מנ"ו דינרי' שיהיה נ"ו דברי' מדינרי‫' | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{67\sdot\left(6-x\right)=402-67x}}</math> | ||
| + | |style="text-align:right;"|ולקחת ו' אמות פחות דבר אחד מס"ז דינרי' שעולה ת"ב דינרי' פחות ס"ז דינרי‫' | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{56x+\left(402-67x\right)=370}}</math> | ||
| + | |style="text-align:right;"|אשר הם שוים אל ש"ע | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ועתה תשוב אל הכלל האמור למעלה שהוא להוציא המספר הקטון מהגדול ולהוסיף על הגורע | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{402-370=32}}</math> | ||
| + | |style="text-align:right;"|ולכן אנו נוציא המספר הקטן מהגדול שהוא ש"ע מת"ב וישאר ל"ב | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{67x-56x=11x}}</math> | ||
| + | |style="text-align:right;"|ועתה {{#annot:term|181,1192|V6IU}}תגרע ה{{#annotend:V6IU}}דברי' הכמות הקטון מהגדול שהוא נ"ו מס"ז וישארו י"א דברים שהם יהיה המחלק | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{a=x=\frac{32}{11}=2+\frac{10}{11}}}</math> | ||
| + | |style="text-align:right;"|רצוני לחלק מספר ל"ב על י"א דברים שעולה ב' וי' חלקים מי"א<br> | ||
| + | וכן הוא אחד מהכמויו' מהבגד | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{b=6-x=3+\frac{1}{11}}}</math> | ||
| + | |style="text-align:right;"|והחלק האחר אם כן הוא {{#annot:term|184,2454|hLvJ}}השארית עד{{#annotend:hLvJ}} ו' שהוא ג' וא' מחלק י"א | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ואשר הוא ב' אמות וי' חלקים מי"א הוא מנ"ו וי' האמה | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ואשר הוא ג' אמות וחלק אחד מי"א הוא מס"ז וי' האמה | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|שעולה סכומם ש"ע דינרים שעושה היטב החשבון שהושם למעלה בעבור הפרק האמור | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ואזכירך כי זה החשבון ממש תוכל לעשותו מן שתי ההנחות כמו שתמצא בזה הספר אם תחפש היטב | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|והנה החשבון האמור נשלם בעבור הפרק הראשון מאלגיבלי | ||
| + | |- | ||
| + | | | ||
| + | *Question: find me a number such that if you divide its third by an eighth it the result is five. | ||
| + | :<math>\scriptstyle\frac{\frac{1}{3}a}{\frac{1}{8}}=5</math> | ||
| + | |style="text-align:right;"|עוד בעבור הפרק הראשון שאלה תמצא לי מספר אחד אשר אם תחלק שלישיתו בשמינית אחד ממספר יעלה ה‫' | ||
| + | |- | ||
| + | | | ||
| + | :defining the number as a thing: <math>\scriptstyle{\color{blue}{a=x}}</math> | ||
| + | |style="text-align:right;"|תניח שהמספר יהיה דבר אחד | ||
| + | |- | ||
| + | | | ||
| + | :*<math>\scriptstyle\frac{\frac{1}{3}x}{\frac{1}{8}}=5</math> | ||
| + | |style="text-align:right;"|תקח שלישיתו שהוא שלישית דבר ו{{#annot:term|784,1259|Nfe3}}חלקהו ב{{#annotend:Nfe3}}שמינית מספר | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ידוע הוא כי כאשר נחלק כמות מה בחלוק מה ו{{#annot:term|783,1223|TQpW}}מספר החלוק העולה{{#annotend:TQpW}} יכפל אחר זה במחלק יגיע מזה אותו הכמות שנחלק ראשונה | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|וכן אם תחלק שליש אחד מדבר בשמינית אחד ממספר ראוי שיגיע ה' תכה א"כ ה' בשמינית אחד וראוי שיגיע שלישית דבר ואם תכפול ה' בשמינית אחד יעשה ה' שמיניות שיהיו שוים לשלישית אחד מדבר ובזה יהיה לך דבר שוה למספר שהוא שלישית דבר שוה לה' שמיניות ממספר שהוא זאת ההשואה מהפרק הראשון א"כ תחלק המספר על הדבר וזהו ה' שמיניות בשלישית אחד מדבר ובזה יהיה לך דבר שוה למספר ויגיע לך ט"ו שמיניות שהם א' וז' שמניות | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ועתה נסה נא תקח השלישית מא' וז' שמיניות ויהיו ה' שמיניות ותחלק בשמינית אחד ויעלה לך היטב ה' ונעשה בעבור הפרק הראשון | ||
| + | |- | ||
| + | | | ||
| + | *Question: three fuzi and one pair of sandales are worth 32 dinar; 6 fuzi and 3 pairs of sandales are worth 80 dinar. | ||
| + | :I ask: how much is one fuzi worth, and how much is one pair of sandals worth? | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle3a+b=32\\\scriptstyle6a+3b=80\end{cases}</math> | ||
| + | |style="text-align:right;"|עוד בעבור הפרק הראשון שאלה ג' פוזי וא' זוג סנדלים שוים ל"ב דינרים<br> | ||
| + | וו' פוזי וג' זוגות סנדלים שוים פ' דינרים<br> | ||
| + | אשאל כמה שוה אחד פוזו וכמה שוה זוג אחד מהסנדלים | ||
| + | |- | ||
| + | | | ||
| + | :defining: the fuzo is worth a thing <math>\scriptstyle{\color{blue}{a=x}}</math> | ||
| + | |style="text-align:right;"|תניח שהפוזו שוה דבר אחד | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|א"כ ג' פוזי שוים ג' דברי' וזוג סנדלי' שוים ל"ב דינרי' פחות ג' דברי' ואח"כ ו' פוסו שוים ו' דברים וג' זוגו' סנדלים שוים צ"ו דינרי' פחות ט' דברים כאשר הנחת למעלה וזה יהיה שוה לפ' דינרי' {{#annot:term|865,1246|zMtq}}תשוה ה{{#annotend:zMtq}}חלקי' תוציא פ' מצ"ו וישאר י"ו עתה הנך יודע כי {{#annot:term|178,1165|2ZJa}}בחבר יחד{{#annotend:2ZJa}} ו' דברי' וצ"ו דינרי' פחות ט' דברי' עושים צ"ו פחות ג' דברים שהם שוי' אל פ' א"כ תחלקם כאמור למעלה ותוציא פ' מצ"ו ישאר י"ו אח"כ תתן לכל חלק ג' דברים מפני כי צריך תמיד {{#annot:term|866,1268|m7fq}}לשלם במקום שיחסר{{#annotend:m7fq}} כאשר הראית למעלה וכמו שאתה רואה בזה החשבון ובזה יהיה לך ג' דברים שוים לי"ו מספרי' תחלק א"כ המספרים על הדברים ויהיה לך ה' ושליש וככה שוה הדבר ואתה הנחת שהפוזו היה שוה דבר אחד א"כ יהיה שוויו ה' דברי' דינרי' ושליש והסנדלי' צריך שישוו בהכרח י"ו דינרי‫' | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|והנה נעשה מן הפרק הנזכ' עוד בעבור הפרק האמור | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:a+b=10, b/a=5|619|xcyr}}Divide ten into two parts, such that when we multiply the one by the other it yields five. | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle a+b=10\\\scriptstyle\frac{b}{a}=5\end{cases}</math> | ||
| + | |style="text-align:right;"|עשה לי מעשרה שני חלקי' אשר כשנחלק האחד באחר יעשה חמשה{{#annotend:xcyr}} | ||
| + | |- | ||
| + | | | ||
| + | :defining: | ||
| + | :*one of the parts as a thing <math>\scriptstyle{\color{blue}{a=x}}</math> | ||
| + | |style="text-align:right;"|תניח שאחד מהחלקים יהיה דבר אחד | ||
| + | |- | ||
| + | | | ||
| + | :*the other should be ten minus a thing <math>\scriptstyle{\color{blue}{b=10-x}}</math> | ||
| + | |style="text-align:right;"|והאחר צריך שיהיה עשרה פחות דבר אחד | ||
| + | |- | ||
| + | | | ||
| + | :*<math>\scriptstyle{\color{blue}{\frac{10-x}{x}=5}}</math> | ||
| + | |style="text-align:right;"|עתה תחלק עשרה פחות דבר אחד על דבר אחד ויגיע ה‫' | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{10-x=5x}}</math> | ||
| + | |style="text-align:right;"|א"כ אם תכפול ה' בדבר אחד יעשה ה' דברי' וזהו שוה אל עשרה פחות דבר אחד | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{10-x+x=5x+x}}</math> | ||
| + | |style="text-align:right;"|{{#annot:term|866,2563|g7ov}}להשחית החוב{{#annotend:g7ov}} רצו' {{#annot:term|866,1268|52ZN}}לשלם במקום החסר{{#annotend:52ZN}} נתן לכל חלק ה{{#annot:term|789,1514|7vBD}}חסרון{{#annotend:7vBD}} שהוא דבר אחד | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{10=6x}}</math> | ||
| + | |style="text-align:right;"|ויהיה לנו עשרה מספרי' ויהיה לנו עשרה בלי חשבון והאחר שהוא ו' דברים יהיה ו' דברי‫'<br> | ||
| + | והנה כי יש לנו שעשרה שוה לו' דברים | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|א"כ נרדוף הכלל ונחלק עשרה על ו' ויהיה העולה א' וב' שלישיו' וככה שוה הדבר ואנחנו הנחנו שהראשון היה דבר אחד א"כ היה א' וב' שלישיו' | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|והחלק האחר היה הנשאר עד עשרה שהוא ח' ושליש | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ואם רצית לנסותו תחלק ח' ושליש על א' וב' שלישי' והיטב יצא ה' ונעשה בעבור הפרק הראשון | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== Chapter Two ==== | ||
| + | |||
| + | |style="width:45%;text-align:right;"|<big>פרק שני</big> | ||
| + | |- | ||
| + | |Its nature is as written right below | ||
| + | |style="text-align:right;"|וטבעו כמו שכתו' תחת זה מיד | ||
| + | |- | ||
| + | |<math>\scriptstyle ax^2=c</math> | ||
| + | |style="text-align:right;"|כאשר הצינסי יהיו שוים אל המספרים | ||
| + | |- | ||
| + | |<math>\scriptstyle x^2=\frac{c}{a}</math> | ||
| + | |style="text-align:right;"|צריך לחלק המספרים על הצינסי והעולה יהיה מספר וככה שוה הצינסי | ||
| + | |- | ||
| + | |<math>\scriptstyle x=\sqrt{\frac{c}{a}}</math> | ||
| + | |style="text-align:right;"|ושרש העולה מזה כן ישוה הדבר | ||
| + | |- | ||
| + | | | ||
| + | *Question: do this calculation for me - two people who have an amount of ten drama, I am not telling you how much does each has separately, but when the drama of both are multiplied by themselves, they make 20¼. | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle a+b=10\\\scriptstyle\left(a-b\right)^2=20+\frac{1}{4}\end{cases}</math> | ||
| + | |style="text-align:right;"|שאלה עשה לי זה החשבון הנה ב' אנשי' אשר להם עשרה דראמ' מעות ואיני אומ' לך כמה דראמ' יש לכל אחד לבדו אבל כשהוכפלו הדרמ' אשר לאחד ולאחר בעצמם יעשו כ' ורביע | ||
| + | |- | ||
| + | |Following the rule: | ||
| + | |style="text-align:right;"|תעשה כמו שאומ' הכלל | ||
| + | |- | ||
| + | | | ||
| + | :defining according to the algebra: | ||
| + | :*one of the parts as a thing plus five numbers <math>\scriptstyle{\color{blue}{a=x+5}}</math> | ||
| + | |style="text-align:right;"|תניח כפי {{#annot:term|132,1825|NLdc}}אלגיברא{{#annotend:NLdc}} שאחד מהחלקים יהיה דבר אחד וה' מספרים | ||
| + | |- | ||
| + | | | ||
| + | :*the other part remains five minus a thing <math>\scriptstyle{\color{blue}{b=5-x}}</math> | ||
| + | |style="text-align:right;"|והחלק האחר ישאר ה' פחות דבר אחד | ||
| + | |- | ||
| + | | | ||
| + | ::The difference between one part and the other: <math>\scriptstyle{\color{blue}{a-b=\left(x+5\right)-\left(5-x\right)=2x}}</math> | ||
| + | |style="text-align:right;"|עתה אקח ההבדל שיש בין החלק האחד לאחר רצו' מדבר אחד וה' מספרים אל דבר אחד פחות ה' מספרים פחות דבר אחד וזה {{#annot:term|997,1651|1e2w}}ההבדל{{#annotend:1e2w}} יהיה ב' דברי‫' | ||
| + | |- | ||
| + | | | ||
| + | ::The reason for this: | ||
| + | |style="text-align:right;"|וסבת זה | ||
| + | |- | ||
| + | | | ||
| + | ::*one of the parts is <math>\scriptstyle{\color{blue}{a=x+5}}</math> | ||
| + | |style="text-align:right;"|כי אחד מהחלקי' הוא דבר אחד וה' מספרים | ||
| + | |- | ||
| + | | | ||
| + | ::*the other part is <math>\scriptstyle{\color{blue}{b=5-x}}</math> | ||
| + | |style="text-align:right;"|והחלק האחר הוא ה' פחות דבר אחד | ||
| + | |- | ||
| + | | | ||
| + | ::therefore, one of the parts exceeds the other by two [things] | ||
| + | |style="text-align:right;"|א"כ אחד מהחלקי' הוא ב' חלקי' יותר מהאחר | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{a-b=\left(x+5\right)-\left(5-x\right)=2x}}</math> | ||
| + | |style="text-align:right;"|ואם תרצה אראך זה באופן אחר בהוציאך ה' פחות דבר אחד מה' ודבר אחד ואם וישאר ב' דברי' וככה הוא הדרמ' האמור דהיינו כמה הוא החלק האחד גדול מהאחר | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{\left(2x\right)^2=4x^2}}</math> | ||
| + | |style="text-align:right;"|וזאת הדרמ' רצוני הב' דברי' תכפלהו בעצמו יעלה ד' צינסי | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle4x^2=20+\frac{1}{4}</math> | ||
| + | |style="text-align:right;"|ואלו הד' צינסי הם שוים אל כ' ורביע | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{x^2=\frac{20+\frac{1}{4}}{4}=5+\frac{1}{16}}}</math> | ||
| + | |style="text-align:right;"|עתה תחלק המספרי' על הצינסי שהם כ' ורביע על ד' ויעלה ה' וחלק אחד מי"ו וככה שוה הצינסי | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{x=\sqrt{5+\frac{1}{16}}=2+\frac{1}{4}}}</math> | ||
| + | |style="text-align:right;"|ושרש ה' וחלק מי"ו הוא הדבר וזה השרש הוא ב' ורביע | ||
| + | |- | ||
| + | | | ||
| + | :You assumed one of the parts is one thing and 5 numbers, so it becomes 2 and a quarter plus 5; the result is 7 and a quarter and this is one of the parts. | ||
| + | :<math>\scriptstyle{\color{blue}{a=x+5=\left(2+\frac{1}{4}\right)+5=7+\frac{1}{4}}}</math> | ||
| + | |style="text-align:right;"|ואתה הנחת שאחד מהחלקי' היה דבר אחד וה' מספרי' א"כ יגיע להיות ב' ורביע וה' שעולה ז' ורביע וככה הוא אחד מהחלקי‫' | ||
| + | |- | ||
| + | | | ||
| + | :The other part is assumed to be 5 minus a thing, which is 5 minus 2 and a quarter; 2 and 3-quarters remain and this is the other part of ten. | ||
| + | :<math>\scriptstyle{\color{blue}{b=5-x=5-\left(2+\frac{1}{4}\right)=2+\frac{3}{4}}}</math> | ||
| + | |style="text-align:right;"|והחלק האחר הונח ה' פחות דבר אחד שהוא ה' פחות ב' ורביע וישאר ב' וג' רביעי' וככה הוא החלק האחד מעשרה | ||
| + | |- | ||
| + | | | ||
| + | :<math>\scriptstyle{\color{blue}{\begin{cases}\scriptstyle a=7+\frac{1}{4}\\\scriptstyle b=2+\frac{3}{4}\end{cases}}}</math> | ||
| + | |style="text-align:right;"|א"כ יהיה לך אחד מהחלקים ז' ורביע והאחר ב' וג' רביעי‫' | ||
| + | |- | ||
| + | | | ||
| + | :Solved according to the [rule] of chapter two | ||
| + | |style="text-align:right;"|ונעשה עם הפר' הב‫' | ||
| + | |- | ||
| + | |Although this calculation was defined by the first method of the algebra, it was not defined by the general conventional method, as the conventional method defines the unknown as one thing, while here it was defined as a thing plus five numbers. | ||
| + | |style="text-align:right;"|הנני מודיעך כי אע"פ שזה החשבון הונח באופן הראשון מהאלזיבר' לא הונח באופן הכללי הנהוג בהיות כי הכללי הנהוג מניח אותו שאינו ידוע דבר אחד ואנחנו הנחנו דבר אחד וה' מספרי' בעשות מעשרה אותם שני חלקים שנשאלו למעלה | ||
| + | |- | ||
| + | |The reason for that is: | ||
| + | |style="text-align:right;"|וסבת זה <sup>למה</sup> היא זו | ||
| + | |- | ||
| + | | | ||
| + | :if defining: | ||
| + | :*one of the parts of ten as a thing <math>\scriptstyle{\color{blue}{a=x}}</math> | ||
| + | |style="text-align:right;"|כי בהניח אחד מהחלקים מעשרה שהיה דבר אחד | ||
| + | |- | ||
| + | | | ||
| + | :*the other part as ten minus a thing <math>\scriptstyle{\color{blue}{b=10-x}}</math> | ||
| + | |style="text-align:right;"|והחלק האחר היה עשרה פחות דבר אחד | ||
| + | |- | ||
| + | | | ||
| + | :then the one part exceeds the other by <math>\scriptstyle{\color{blue}{b-a=\left(10-x\right)-x=10-2x}}</math> | ||
| + | |style="text-align:right;"|והדרמ' היתה אז פחות ב' דברים מפני כי להוציא דבר אחד מעשרה פחות דבר אחד ישאר עשרה פחות ב' דברים וככה הוא הדרמ' רצוני אשר הוא החלק האחד הגדול מחבירו | ||
| + | |- | ||
| + | | | ||
| + | :and by pursuing [the rule] according to the said conditions, the calculation is necessarily restored to chapter five. | ||
| + | |style="text-align:right;"|וברצות לרדוף בחשבון כפי התנאים האמורי' להשיבו אל התכלית יבא אל הפרק הה' בהכרח | ||
| + | |- | ||
| + | | | ||
| + | :Since the wish was to restore it to chapter two, it was defined as a thing plus five <math>\scriptstyle{\color{blue}{a=x+5}}</math> as shown above. | ||
| + | |style="text-align:right;"|ואנחנו רצינו להשיבו בפרק השני ולכן שמנו אל דבר אחד וה' מספרים כאשר הראה למעלה | ||
| + | |- | ||
| + | | | ||
| + | :If the other part is subtracted from the first, the remainder is <math>\scriptstyle{\color{blue}{a-b=x-\left(10-x\right)=2x-10}}</math> | ||
| + | |style="text-align:right;"|עוד תדע כי אם רצית להוציא החלק האחר שהוא עשרה פחות דבר אחד חוצה מהחלק האחר האמור דבר אחד יהיה הנשאר ב' דברים פחות עשרה והיית יכול לאמר שזה יהיה ההבדל באמת | ||
| + | |- | ||
| + | | | ||
| + | :by pursuing [the rule], the calculation is also restored to chapter five. | ||
| + | |style="text-align:right;"|ולרדוף עוד בזה האופן הרושם החשבון יצא גם כן מהפרק החמישי | ||
| + | |- | ||
| + | |The things [<math>\scriptstyle{\color{blue}{x}}</math>] would have been derived differently, but the parts [<math>\scriptstyle{\color{blue}{a}}</math>; <math>\scriptstyle{\color{blue}{b}}</math>] would have been well defined, as will be shown below in chapter five. | ||
| + | |style="text-align:right;"|אבל הדברים היו באים שונים זה מזה והחלקי' היו באים בזה עשויים היטב כפי מה שנראך בדרכינו לפנים בפרק הה‫' | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|בהיות כי מהפרק האמור יענה פעמים רבות חשבונות מה מספרים ושרשים וחשבונו' מה מספרי' פחות שרשים ואחרי' יוכלו לענות יותר שרשי' ואחדים פחות שרשים ונעשה מהפרק השני | ||
| + | |- | ||
| + | |Another one of chapter two: | ||
| + | |style="text-align:right;"|עוד מהפרק השני | ||
| + | |- | ||
| + | | | ||
| + | *Find me a number such that when multiplied by itself, then the half of that number is multiplied by itself, and you sum the two products together, it makes ten. | ||
| + | :<math>\scriptstyle a^2+\left(\frac{1}{2}a\right)^2=10</math> | ||
| + | |style="text-align:right;"|תמצא לי מספר אחד כי כשהוכה בעצמו ואח"כ מחצית אותו מספר יוכה בעצמו ואלו שתי ההכפלו' תחבר יחד יעשה עשרה | ||
| + | |- | ||
| + | | | ||
| + | :defining the number as a thing <math>\scriptstyle{\color{blue}{a=x}}</math> | ||
| + | |style="text-align:right;"|תניח כי המספר יהיה דבר אחד | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{\left(x\right)^2=x^2}}</math> | ||
| + | |style="text-align:right;"|תכפלהו בעצמו ועושה צינסו אחד | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{\left(\frac{1}{2}x\right)^2=\frac{1}{4}x^2}}</math> | ||
| + | |style="text-align:right;"|אח"כ תכפול מחצית הדבר ויעשה רביע אחד מצינסו | ||
| + | |- | ||
| + | | | ||
| + | ::::<math>\scriptstyle{\color{blue}{x^2+\frac{1}{4}x^2=\left(1+\frac{1}{4}\right)x^2}}</math> | ||
| + | ::<math>\scriptstyle\left(1+\frac{1}{4}\right)x^2=10</math> | ||
| + | |style="text-align:right;"|תחברם יחד ויעשה צינסו אחד ורביע אחד מצינסו והוא שוה לעשרה | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{x^2=\frac{10}{1+\frac{1}{4}}=9}}</math> | ||
| + | |style="text-align:right;"|עתה תחלק המספר על הצינסו דהיינו עשרה על א' צינסו ורביע ויעלה שרש ח‫' | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{x=\sqrt{8}}}</math> | ||
| + | |style="text-align:right;"|ושורש ח' ישוה הדבר ואתה הנחת שהמספר היה דבר אחד א"כ היה שרש ח‫' | ||
| + | |- | ||
| + | |Solved according to chapter two. | ||
| + | |style="text-align:right;"|ונעשה מהפרק השני | ||
| + | |- | ||
| + | |Another one of chapter two: | ||
| + | |style="text-align:right;"|עוד מהפרק השני | ||
| + | |- | ||
| + | | | ||
| + | *Find me a number such that when multiplied by its three quarters it makes forty. | ||
| + | :<math>\scriptstyle a\sdot\frac{3}{4}a=40</math> | ||
| + | |style="text-align:right;"|תמצא לי מספר אחד שכאשר הוכה על ג' רביעיו יעשה מ‫' | ||
| + | |- | ||
| + | |Following the rule: | ||
| + | |style="text-align:right;"|תעשה כמו שאומ' הכלל | ||
| + | |- | ||
| + | | | ||
| + | :defining the number as a thing <math>\scriptstyle{\color{blue}{a=x}}</math> | ||
| + | |style="text-align:right;"|תניח שהמספר יהיה דבר אחד | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{\left(\frac{3}{4}x\right)\sdot x=\frac{3}{4}x^2}}</math> | ||
| + | ::<math>\scriptstyle\frac{3}{4}x^2=40</math> | ||
| + | |style="text-align:right;"|תקח ג' רביעיו שהם ג' רביעים מדבר תכפלם בדבר אחד ועושה ג' רביעים מצינסו שהם שוים אל מ' מספרי‫' | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{x^2=\frac{40}{\frac{3}{4}}=53+\frac{1}{3}}}</math> | ||
| + | |style="text-align:right;"|תחלק המספרי' על הצינסי דהיינו מ' על ג' רביעי' ויגיע לך נ"ג ושליש | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{x=\sqrt{53+\frac{1}{3}}}}</math> | ||
| + | |style="text-align:right;"|וכן שורש נ"ג ושליש שוה הדבר ואתה הנחת שהמספר היה דבר אחד א"כ היה שרש נ"ג ושליש | ||
| + | |- | ||
| + | |This number is inexpressible | ||
| + | |style="text-align:right;"|וזה המספר הוא בלתי מדבר ואי אפש' לבטאות בו | ||
| + | |- | ||
| + | |Solved according to chapter two. | ||
| + | |style="text-align:right;"|ונעשה מהפרק השני | ||
| + | |- | ||
| + | |Another one of chapter two: | ||
| + | |style="text-align:right;"|עוד מהפרק השני | ||
| + | |- | ||
| + | | | ||
| + | *Find me a number such that when its third and its quarter are subtracted from it, and the remainder is multiplied by itself it makes twelve. | ||
| + | :<math>\scriptstyle\left(a-\frac{1}{3}a-\frac{1}{4}a\right)^2=12</math> | ||
| + | |style="text-align:right;"|תמצא לי מספר אחד אשר כשהוצא ממנו שלישיתו ורביעיתו והנשאר יוכה בעצמו יעשה י"ב | ||
| + | |- | ||
| + | | | ||
| + | :defining the number as a thing <math>\scriptstyle{\color{blue}{a=x}}</math> | ||
| + | |style="text-align:right;"|תניח שהמספר היה דבר אחד | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{\frac{1}{3}x+\frac{1}{4}x=\frac{7}{12}x}}</math> | ||
| + | |style="text-align:right;"|והשליש והרביע מדבר הוא ז' חלקים מי"ב | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{x-\frac{7}{12}x=\frac{5}{12}x}}</math> | ||
| + | |style="text-align:right;"|הוציאם מדבר אחד ישאר ה' חלקים מי"ב מדבר | ||
| + | |- | ||
| + | | | ||
| + | ::::<math>\scriptstyle{\color{blue}{\left(\frac{5}{12}x\right)^2=\frac{25}{144}x^2}}</math> | ||
| + | ::<math>\scriptstyle\frac{25}{144}x^2=12</math> | ||
| + | |style="text-align:right;"|עתה תכפול ה' חלקים מי"ב בדבר על ה' חלקי' מי"ב בדבר ועושה כ"ה חלקים מקמ"ד בצינסו שהם שוים אל י"ב | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{x^2=\frac{12}{\frac{25}{144}}=63+\frac{3}{25}}}</math> | ||
| + | |style="text-align:right;"|א"כ תחלק י"ב על כ"ה חלקים מקמ"ד בצינסו ויגיע לך ס"ט וג' חלקים מכ"ה | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{x=\sqrt{63+\frac{3}{25}}}}</math> | ||
| + | |style="text-align:right;"|ושרשו ישוה הדבר ואתה הנחת שהמספר היה דבר אחד א"כ היה שרש מס"ט וג' חלקים מכ"ה | ||
| + | |- | ||
| + | |Solved according to chapter two. | ||
| + | |style="text-align:right;"|ונעשה מהפרק השני | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== Chapter Three ==== | ||
| + | |||
| + | |style="width:45%;text-align:right;"|<big>הפרק השלישי</big> | ||
| + | |- | ||
| + | |<math>\scriptstyle ax^2=bx</math> | ||
| + | |style="text-align:right;"|וטבעו כאשר הצינס' יהיו שוים לדברים | ||
| + | |- | ||
| + | |<math>\scriptstyle x=\frac{b}{a}</math> | ||
| + | |style="text-align:right;"|צריך לחלק הדברים בצינסי ו{{#annot:term|876,2086|Wi1E}}המגיע{{#annotend:Wi1E}} הוא מספר<br> | ||
| + | וכך שוה הדבר | ||
| + | |- | ||
| + | | | ||
| + | *find me a number such that when multiplied by its two thirds makes three times the number | ||
| + | :<math>\scriptstyle a\sdot\frac{2}{3}a=3a</math> | ||
| + | |style="text-align:right;"|תמצא לי מספר אחד אשר כשהוכה בשני שלישיו יעשה ג' פעמי' כמו המספר הנמצא | ||
| + | |- | ||
| + | |Following its rule: | ||
| + | |style="text-align:right;"|תעשה כמו שאומ' הכלל שלו | ||
| + | |- | ||
| + | | | ||
| + | :defining the number as a thing <math>\scriptstyle{\color{blue}{a=x}}</math> | ||
| + | |style="text-align:right;"|תניח שהמספר יהיה דבר אחד | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{\frac{2}{3}x}}</math> | ||
| + | |style="text-align:right;"|ועתה תקח שני שלישיו שהוא ב' שלישי דבר | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{\left(\frac{2}{3}x\right)\sdot x=\frac{2}{3}x^2}}</math> | ||
| + | |style="text-align:right;"|ותכה ב' שלישי דבר בדבר ועולה ב' שלישי צינסו | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle3x=\frac{2}{3}x^2</math> | ||
| + | |style="text-align:right;"|ואלו ב' שלישי צינסו הם שוים לשלשה פעמים המספר הנמצא דהיינו ג' דברים שוים לב' שלישי צינסו | ||
| + | |- | ||
| + | | | ||
| + | :According the aforesaid rule: dividing the things by the çenso | ||
| + | |style="text-align:right;"|אשר אנו צריכי' לחלק כפי הכלל האמור למעלה שהוא לחלק הדברים על הצינסי | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{x=\frac{3}{\frac{2}{3}}=4+\frac{1}{2}}}</math> | ||
| + | |style="text-align:right;"|שהוא ג' בב' שלישיו שיגיע ממנו ד' וחצי וככה שוה הדבר רצוני המספר הנשאל | ||
| + | |- | ||
| + | |Another one of the said chapter: | ||
| + | |style="text-align:right;"|עוד מהפרק האמור | ||
| + | |- | ||
| + | | | ||
| + | *find me two numbers such that the one is part of the other as 2 are to 3, and the product of the one by the other is the same as their sum together. | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle a:b=2:3\\\scriptstyle a\sdot b=a+b\end{cases}</math> | ||
| + | |style="text-align:right;"|תמצא לי ב' מספרים שיהיה האחד חלק מהאחר כמו שהוא ב' מג‫'<br> | ||
| + | ויעשה כשהוכו האחד באחר כמו כאשר חוברו יחד | ||
| + | |- | ||
| + | | | ||
| + | :defining: | ||
| + | :*the first as two things <math>\scriptstyle{\color{blue}{a=2x}}</math> | ||
| + | |style="text-align:right;"|תניח שהראשון היה ב' דברים | ||
| + | |- | ||
| + | | | ||
| + | :*the second as three things <math>\scriptstyle{\color{blue}{b=3x}}</math> | ||
| + | |style="text-align:right;"|והשני ג' דברים | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{2x+3x=5x}}</math> | ||
| + | |style="text-align:right;"|עתה תחבר ב' דברי' עם ג' דברים ועושים ה' דברים | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{2x\sdot3x=6x^2}}</math> | ||
| + | |style="text-align:right;"|עתה תכה ב' דברי' בג' דברי' ועושים ו' צינסי | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle5x=6x^2</math> | ||
| + | |style="text-align:right;"|עתה יש לנו שה' דברים הם שוים לו' צינסו | ||
| + | |- | ||
| + | | | ||
| + | :According the aforesaid rule: dividing the things by the çenso | ||
| + | |style="text-align:right;"|תשוב אל הכלל האמור למעלה שהוא לחלק הדברי' על הצינסי | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{x=\frac{5}{6}}}</math> | ||
| + | |style="text-align:right;"|דהיינו ה' על ו' שעולה ה' ששיות וככה שוה הדבר | ||
| + | |- | ||
| + | | | ||
| + | :You assumed the first number is 2 things, so it is 1 and 2-thirds. | ||
| + | :<math>\scriptstyle{\color{blue}{a=2x=1+\frac{2}{3}}}</math> | ||
| + | |style="text-align:right;"|ואתה הנחת שהמספר הראשון היה ב' דברי' א"כ היה א' וב' שלישי‫' | ||
| + | |- | ||
| + | | | ||
| + | :You assumed also the second is 3 things, so 3 times 5-sixths is 2 and a half and this is the second number. | ||
| + | :<math>\scriptstyle{\color{blue}{b=3x=3\sdot\frac{5}{6}=2+\frac{1}{2}}}</math> | ||
| + | |style="text-align:right;"|ועוד הנחת שהשני היה ג' דברי' א"כ היה ג' פעמי' ה' ששיות שעולים ב' וחצי וככה היה המספר השני | ||
| + | |- | ||
| + | |Solved according to chapter three. | ||
| + | |style="text-align:right;"|ונעשה מהפרק השלישי | ||
| + | |- | ||
| + | |Another one of chapter three: | ||
| + | |style="text-align:right;"|עוד מהפרק השלישי | ||
| + | |- | ||
| + | | | ||
| + | *find me a number such that its half multiplied by itself makes the same as the number multiplied by twenty. | ||
| + | :<math>\scriptstyle\left(\frac{1}{2}a\right)^2=20a</math> | ||
| + | |style="text-align:right;"|תמצא לי מספר אחד ש{{#annot:term|845|sDfL}}מחציתו{{#annotend:sDfL}} מוכה בעצמו יעשה כמו אם הוכה המספר בעשרים | ||
| + | |- | ||
| + | | | ||
| + | :defining the the number as a thing <math>\scriptstyle{\color{blue}{a=x}}</math> | ||
| + | |style="text-align:right;"|תניח שהיה המספר דבר אחד | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{\frac{1}{2}x}}</math> | ||
| + | |style="text-align:right;"|תקח מחציתו שהוא חצי דבר | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{\left(\frac{1}{2}x\right)^2=\frac{1}{4}x^2}}</math> | ||
| + | |style="text-align:right;"|תכהו בעצמו ועושה רביע צינסו | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{x\sdot20=20x}}</math> | ||
| + | ::<math>\scriptstyle20x=\frac{1}{4}x^2</math> | ||
| + | |style="text-align:right;"|אח"כ תכפול דבר אחד בעשרים ועושה כ' דברים שהם שוים לרביע אחד מצינסו | ||
| + | |- | ||
| + | | | ||
| + | :Dividing the things by the çenso | ||
| + | |style="text-align:right;"|ועתה תחלק הדברים על הצינסי | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{a=x=\frac{20}{\frac{1}{4}}=80}}</math> | ||
| + | |style="text-align:right;"|ויעלה פ' וככה שוה הדבר ואתה הנחת שהמספר היה דבר אחד א"כ היה הוא פ‫' | ||
| + | |- | ||
| + | |Solved according to chapter three. | ||
| + | |style="text-align:right;"|ונעשה מהפרק השלישי | ||
| + | |- | ||
| + | |Another one of chapter three: | ||
| + | |style="text-align:right;"|עוד מהפרק השלישי | ||
| + | |- | ||
| + | | | ||
| + | *find me a number such that its product by itself makes the same as the number divided by a hundred. | ||
| + | :<math>\scriptstyle a^2=\frac{a}{100}</math> | ||
| + | |style="text-align:right;"|תמצא לי מספר אחד שיעשה הכאתו בעצמו כל כך שעושה אם נחלק על ק‫' | ||
| + | |- | ||
| + | | | ||
| + | :defining the the number as a thing <math>\scriptstyle{\color{blue}{a=x}}</math> | ||
| + | |style="text-align:right;"|תניח שהמספר היה דבר אחד | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{\left(x\right)^2=x^2}}</math> | ||
| + | |style="text-align:right;"|תכהו בעצמו ועושה צינסו אחד | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{\frac{x}{100}=\frac{1}{100}x}}</math> | ||
| + | ::<math>\scriptstyle\frac{1}{100}x=x^2</math> | ||
| + | |style="text-align:right;"|אח"כ תחלק דבר אחד בק' ויעלה חלק אחד מק' בדבר שהוא שוה לא' צינסו | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{a=x=\frac{\frac{1}{100}}{1}=\frac{1}{100}}}</math> | ||
| + | |style="text-align:right;"|תחלק חלק אחד מק' על אחד ויעלה עוד חלק אחד מק' וכך שוה הדבר ואתה הנחת שהיה המספר דבר אחד א"כ היה חלק אחד מק‫' | ||
| + | |- | ||
| + | |Solved according to chapter three. | ||
| + | |style="text-align:right;"|ונעשה מפרק ג‫' | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== Chapter Four ==== | ||
| + | |||
| + | |style="width:45%;text-align:right;"|הפרק הרביעי | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|והוא המורכב ראשון טבעו | ||
| + | |- | ||
| + | |<math>\scriptstyle ax^2+bx=c</math> | ||
| + | |style="text-align:right;"|כאשר הצינסי והדברי' יהיו שוים אל המספר | ||
| + | |- | ||
| + | |<math>\scriptstyle x=\sqrt{\left(\frac{\frac{b}{a}}{2}\right)^2+\frac{c}{a}}-\frac{\frac{b}{a}}{2}</math> | ||
| + | |style="text-align:right;"|צריך לחלק כל ההשואה על הצינסי<br> | ||
| + | אח"כ תחלק מחצית הדברים לחצי<br> | ||
| + | ואחד מאותם החצאים תכהו בעצמו<br> | ||
| + | ואותה ההכאה תחבר על המספר<br> | ||
| + | והשרש מכל הסך פחות מחצית האחר מהדברי' יהיה שווי הדבר | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:a+b=10, a²=¼b|619|r2I0}}Divide ten into two parts, such that the product of the small part by itself is the same as a quarter of the product of the the large [part] by itself | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle a+b=10\\\scriptstyle a^2=\frac{1}{4}b\end{cases}</math> | ||
| + | |style="text-align:right;"|עשה לי מעשרה שני חלקי' שכשהוכה החלק הקטן בעצמו יעשה כל כך כמו שיעשה הכאת רביע הגדול בעצמו{{#annotend:r2I0}} | ||
| + | |- | ||
| + | |Following the rule: | ||
| + | |style="text-align:right;"|תעשה כמו שאומ' הכלל | ||
| + | |- | ||
| + | | | ||
| + | :defining: | ||
| + | :*one of the parts as a thing <math>\scriptstyle{\color{blue}{a=x}}</math> | ||
| + | |style="text-align:right;"|תניח שאחד מהחלקי' יהיה דבר אחד | ||
| + | |- | ||
| + | | | ||
| + | :*the other should remain ten minus a thin <math>\scriptstyle{\color{blue}{b=10-x}}</math> | ||
| + | |style="text-align:right;"|והאחר צריך שישאר עשרה פחות דבר אחד | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|אח"כ תוכל להניח אי זה מהם שתרצה בעד הגדול | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ואנחנו נניח כי החלק הקטן יהיה דבר אחד | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|וסבת למה היא זאת אם הנחת שהחלק הגדול היה דבר אחד היה יוצא החשבון אל הפרק החמישי מפני ההשואה | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ואנחנו מבקשים להשיבו אל הרביעי אשר אנחנו בו | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{x\sdot x=x^2}}</math> | ||
| + | |style="text-align:right;"|ולכן אנחנו נכה דבר אחד בעצמו בעד החלק הקטן ועושה א' צינסו | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{\frac{1}{4}\sdot\left(10-x\right)=\left(2+\frac{1}{2}\right)-\frac{1}{4}x}}</math> | ||
| + | |style="text-align:right;"|עתה נקח הרביע מהגדול שהוא עשרה פחות דבר אחד ויהיה ב' וחצי פחות רביע אחד מדבר | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{\left[\left(2+\frac{1}{2}\right)-\frac{1}{4}x\right]^2=\left(6+\frac{1}{4}\right)+\frac{1}{16}x^2-\left(1+\frac{1}{4}\right)x}}</math> | ||
| + | |style="text-align:right;"|וזה כאשר הוכה בעצמו עולה ששה מספרים ורביע וחלק א' מי"ו בצינסו פחות דבר אחד ורביע | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(6+\frac{1}{4}\right)+\frac{1}{16}x^2-\left(1+\frac{1}{4}\right)x=x^2}}</math> | ||
| + | |style="text-align:right;"|וזה הכפל הוא שוה לצינס' אחד שהוא כפל החלק הראשון בעצמו | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{x^2-\frac{1}{16}x^2=\frac{15}{16}x^2}}</math> | ||
| + | |style="text-align:right;"|ועתה צריך אתה לרדוף בזה האופן בהיות כי כל אחד מהחלקי' יש לו צינסו הנך צריך להוציא הצינסו שבחלק הקטון מהצינסו שהם בגדול דהיינו להוציא מכל אחד מהחלקי' כל כך צינסו כמו שהוא החלק הקטון וישארו החלקים שוים זה לזה והראשון ישאר ט"ו חלקים מי"ו בצינסו | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|עתה הנך צריך לחבר אל כל אחד מהחלקים כל כך דברים כמו שיש לאחד מהחלקים פחות | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{15}{16}x^2+\left(1+\frac{1}{4}\right)x=\left(6+\frac{1}{4}\right)}}</math> | ||
| + | |style="text-align:right;"|א"כ תחבר לחלק אשר לו דבר אחד ורביע {{#annot:term|789,1364|fBvk}}מפחת{{#annotend:fBvk}} אותו עצמו שיש לו מפחת ויהיה לך אין כלום מפחת ואל החלק האחר תחבר אותו בעצמו שהוא דבר אחד ורביע ויהיה לך אחד מהחלקים בהיות מוצאים הצינסו ומחוברי' הדברים כפי האמור למעלה שוה לאחר רצוני לראשון שהוא ט"ו חלקי' מי"ו בצינסו ודבר אחד ורביע שוה לחלק האחר שהוא ו' ורביע והוא השני | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{15}{16}x^2+\left(1+\frac{1}{4}\right)x=\left(6+\frac{1}{4}\right)\quad/\div\frac{15}{16}}}</math> | ||
| + | |style="text-align:right;"|עתה תחלק כל ההשואה על הצינסו שהוא ט"ו חלקי' מי"ו | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{x^2+\left(1+\frac{1}{3}\right)x=\left(6+\frac{2}{3}\right)}}</math> | ||
| + | |style="text-align:right;"|ויעלה אחד מהחלקים א' צינס' ודבר אחד ושליש שוה לו' מספרי' וב' שלישי' שהם החלק השני | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ועתה אנחנו צריכי' לחלק כפי הכלל האמור קודם הפרק האמור רצו' הדברי' לחצי שיהיו אחד מהחצאים יהיו ב' שלישי‫'<br> | ||
| + | ואלו הב' שלישים צריכי' אנו לכפול בעצמם אשר יעלו ד' תשיעיות<br> | ||
| + | וזה הכפל אנו צריכי' לחבר על המספר רצו' על ו' וב' שלישי' ועולה ז' וא' תשיעית<br> | ||
| + | ושרש ז' וא' תשיעית פחות המחצית האחר מהדברי' דהיינו פחות ב' שלישי' הוא יהיה הדבר שהוא אחד מהחלקי‫' | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle a=x&\scriptstyle=\sqrt{\left[\frac{1}{2}\sdot\left(1+\frac{1}{3}\right)\right]^2+\left(6+\frac{2}{3}\right)}-\left[\frac{1}{2}\sdot\left(1+\frac{1}{3}\right)\right]=\sqrt{\left(\frac{2}{3}\right)^2+\left(6+\frac{2}{3}\right)}-\frac{2}{3}\\&\scriptstyle=\sqrt{\frac{4}{9}+\left(6+\frac{2}{3}\right)}-\frac{2}{3}=\sqrt{7+\frac{1}{9}}-\frac{2}{3}\\\end{align}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{b=10-x=10+\frac{2}{3}-\sqrt{7+\frac{1}{9}}}}</math> | ||
| + | |style="text-align:right;"|והחלק האחר הוא הנשאר עד עשרה שהוא עשרה וב' שלישי' פחות שרש ז' וא' תשיעי‫' | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{\sqrt{7+\frac{1}{9}}=\frac{8}{3}=2+\frac{2}{3}}}</math> | ||
| + | |style="text-align:right;"|ומפני שז' ותשיעית יש לו שרש אשר הוא ח' שלישי' והם ב' וב' שלישי‫' | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{a=x=\sqrt{7+\frac{1}{9}}-\frac{2}{3}=\left(2+\frac{2}{3}\right)-\frac{2}{3}=2}}</math> | ||
| + | |style="text-align:right;"|והאמור ראוי להיות ב' שלישי' פחות מהשרש האמור א"כ תוציא ב' שלישי' מב' וב' שלישי' וישאר ב' וככה הוא החלק הראשון | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{b=10-x=10-2=8}}</math> | ||
| + | |style="text-align:right;"|ואלו הב' תוציא מעשרה וישארו ח' וככה הוא החלק השני | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|עתה רצוני להזכירך שאם השאלה תאמ' שכך עושה החלק הראשון מוכה בעצמו כמו רביע החלק הגדול ה מוכה בעצמו בחלוף מה שאומ' כמו שיעשה הכאת רביע הגדול בעצמו הנה תהיה שאלה אחרת מפני ההבדל אשר יש בין מאמ' למאמ' כי באמרנו רביע הגדול מוכה בעצמו צריך לכפול כל החלק בעצמו וממנו ילקח הרביע ובאמרנו כמו שהוא הכאת רביע הגדול בעצמו צריך לקחת ראשונה החלק הגדול ואותו הרביע תכהו בעצמו וזהו המובן מחשבוננו | ||
| + | |- | ||
| + | | | ||
| + | *Find me a number such that when its product by itself and its product by eight are summed together, the result is 33. | ||
| + | :<math>\scriptstyle a^2+8a=33</math> | ||
| + | |style="text-align:right;"|עוד בעבור הפרק הרביעי תמצא לי מספר אחד שכאשר הוכה בעצמו ואחר יוכה בח' ו{{#annot:term|388,1217|lWWr}}מקובץ{{#annotend:lWWr}} שתי אלו ההכאות יחד יהיה ל"ג | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{a=x}}</math> | ||
| + | |style="text-align:right;"|תניח שהמספר היה דבר אחד | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{x\sdot x=x^2}}</math> | ||
| + | |style="text-align:right;"|תכהו בעצמו עושה א' צינסו | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{x\sdot8=8x}}</math> | ||
| + | |style="text-align:right;"|אח"כ תכהו בח' ועושה ח' דברים | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{x^2+8x=33}}</math> | ||
| + | |style="text-align:right;"|תחברם יחד ויהיה לך א' צינסו וח' דברים שוים אל ל"ג | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|הנך צריך לחלק על הצינסי דהיינו להשיב אל צינסו אחד על א' צינסו ועולה עוד א' צינסו וכיוצא בזה תחלק ח' על אחד ויגיע עוד ח' ותחלק ל"ג על אחד יגיע עוד ל"ג | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|עתה {{#annot:term|786,1369|wzLZ}}תחצה ה{{#annotend:wzLZ}}דברי' שהם ח' ויהיה לך ד‫'<br> | ||
| + | תכהו בעצמו ועושה י"ו<br> | ||
| + | תחברם אל המספר שהוא ל"ג ועושה מ"ט<br> | ||
| + | תקח שרשו ו{{#annot:term|181,1192|VsLC}}תגרע ממנו{{#annotend:VsLC}} מחצית הדברים שהוא ד' והנשאר יהיה שוה לדבר<br> | ||
| + | ואתה הנחת שהמספר היה דבר אחד א"כ היה שרש מ"ט פחות ד' שהוא ג' מספרי‫' | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{x=\sqrt{\left(\frac{1}{2}\sdot8\right)^2+33}-\left(\frac{1}{2}\sdot8\right)=\sqrt{4^2+33}-4=\sqrt{16+33}-4=\sqrt{49}-4=3}}</math> | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|וזה נעשה מהפרק הרביעי שהוא המורכב ראשון | ||
| + | |- | ||
| + | | | ||
| + | *Find me a number such that when the product of its third by itself is added to the number the result is 12. | ||
| + | :<math>\scriptstyle\left(\frac{1}{3}a\right)^2+a=12</math> | ||
| + | |style="text-align:right;"|עוד בעבור הפרק הרביעי תמצא לי מספר אחד שכשהוכה שלישיתו בעצמו ותחובר ההכאה ההיא על המספר יעשה י"ב | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{a=x}}</math> | ||
| + | |style="text-align:right;"|תניח שהמספר יהיה דבר אחד | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{\frac{1}{3}\sdot x=\frac{1}{3}x}}</math> | ||
| + | |style="text-align:right;"|תקח שלישיתו שהוא א' שליש מדבר | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{\left(\frac{1}{3}x\right)^2=\frac{1}{9}x^2}}</math> | ||
| + | |style="text-align:right;"|תכהו בעצמו ועושה א' תשיעית מצינסו | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{1}{9}x^2+x=12}}</math> | ||
| + | |style="text-align:right;"|תחברהו עם דבר אחד ויהיה לנו א' תשיעית מצינסו ודבר אחד שוה אל י"ב | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{1}{9}x^2+x=12\quad/\div\frac{1}{9}}}</math> | ||
| + | |style="text-align:right;"|עתה תחלק הצינסי דהיינו כל החלקים על א' תשיעית | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{x^2+9x=108}}</math> | ||
| + | |style="text-align:right;"|ויהיה לך א' צינסו וט' דברי' וק"ח דראמי דהיינו מספרי' וזאת ההשואה היא א' צינסו וט' דברים שהם שוים אל ק"ח דראמ‫' | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|עתה תחצה הדברי' ויהיה לך ד' וחצי<br> | ||
| + | תכפלם בעצמם ויעלה כ' ורביע<br> | ||
| + | תחברם אל המספרים שהוא ק"ח ויעלו קכ"ח ורביע<br> | ||
| + | תפיל החצי האחד מהדברים מהשרש מקכ"ח ורביע וככה שוה הדבר<br> | ||
| + | ואתה הנחת שהמספר היה דבר אחד א"כ היה שרש קכ"ח ורביע פחות ד' וחצי | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle x&\scriptstyle=\sqrt{\left(\frac{1}{2}\sdot9\right)^2+108}-\left(\frac{1}{2}\sdot9\right)=\sqrt{\left(4+\frac{1}{2}\right)^2+108}-\left(4+\frac{1}{2}\right)=\sqrt{\left(20+\frac{1}{4}\right)+108}-\left(4+\frac{1}{2}\right)\\&\scriptstyle=\sqrt{128+\frac{1}{4}}-\left(4+\frac{1}{2}\right)\\\end{align}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ונעשה מהפרק הרביעי שהוא גם כן המורכב ראשון | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|עוד בעבור הפרק הרביעי אחד הלוה לאחר כ' ליטר' בעד ב' שנים לעשות ראש משנה לשנה ובסוף שתי שנים השיב לו בין קרן וריוח ל' ליטר‫'<br> | ||
| + | אשאל לאי זה חשבון הולוה הליט' לחדש | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|תניח שהולוה לא' דבר ממעו' לחדש | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|וישוו לשנה אחת י"ב דברי‫' | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|תקח א' מעשרים מליט' אחת שהיא היטב כ' דינרים שהוא דינר אחד א"כ נאמ' אנחנו עוד חלק מעשרים כאמור למעלה ויהיה לך כ' ליט' ודבר אחד עתה תקח החלק אחד מעשרים ודבר אחד שהוא דבר אחד וחלק אחד מעשרים בצינסו ויהיה לך סכומם שיהיה כ' ליטר' וב' דברים וחלק אחד מכ' בצינסו שהם שוים אל ל' הוצא כ' מל' וישאר עשרה עתה תחלק על הצינסי כל החלקים ויגיע לך א' צינסו ומ' דברים שיהיו שוים למאתים מספרי‫' | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|א"כ תחצה הדברים שהם מ' ויהיה לך כ‫'<br> | ||
| + | תכפלם בעצמם ויהיה לך ד' מאות<br> | ||
| + | תחברם אל המספר שהוא מאתים ויהיה סכומם ו' מאות<br> | ||
| + | תקח שרשם ותפיל ממנו מחצית הדברים וכן שרש ו' מאות פחות כ' שוה הדבר | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{x=\sqrt{\left(\frac{1}{2}\sdot40\right)^2+200}-\left(\frac{1}{2}\sdot40\right)=\sqrt{20^2+200}-20=\sqrt{400+200}-20=\sqrt{600}-20=8-5}}</math> | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ואתה הנחת שהליטר' הולותה לא' דבר לחדש א"כ הולותה לשרש ו' מאות פחות כ' ממעות לחדש | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|והוא נעשה מן הראשון המורכב | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== Chapter Five ==== | ||
| + | |||
| + | |style="width:45%;text-align:right;"|הפרק החמישי מן ה{{#annot:term|132,1825|8XWM}}אלזיברא{{#annotend:8XWM}} | ||
| + | |- | ||
| + | |We show you its nature in it and it is the second compound [type of equations] | ||
| + | |style="text-align:right;"|נראה לך בו טבעו והוא המורכב השני | ||
| + | |- | ||
| + | |When squares and numbers are equal to things. | ||
| + | :<math>\scriptstyle ax^2+c=bx</math> | ||
| + | |style="text-align:right;"|כאשר הצינסי והמספרים יהיו שוים אל הדברי‫' | ||
| + | |- | ||
| + | |The whole equation should be divided by the number of the squares. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{x=\left(\frac{1}{2}\sdot\frac{b}{a}\right)\pm\sqrt{\left(\frac{1}{2}\sdot\frac{b}{a}\right)^2-\frac{c}{a}}}}</math> | ||
| + | |style="text-align:right;"|צריך לחלק כל ההשואה על כמות הצינסי | ||
| + | |- | ||
| + | |Then, [the number of] the things is divided in half. | ||
| + | |style="text-align:right;"|ואח"כ לחלק הדברים לחצי | ||
| + | |- | ||
| + | |One of the halves is multiplied by itself. | ||
| + | |style="text-align:right;"|ולכפול אחד מן החצאים בעצמו | ||
| + | |- | ||
| + | |This product is subtracted from the number. | ||
| + | |style="text-align:right;"|ולהוציא מן הכפל ההוא המספרים | ||
| + | |- | ||
| + | |Add the root of the remainder to the other half of [the number of] the things and this is equal to the thing. | ||
| + | |style="text-align:right;"|ושרש הנשאר תחבר אל המחצית האחר מהדברי‫'<br> | ||
| + | וככה שוה הדבר | ||
| + | |- | ||
| + | |Sometimes, in certain calculations, when the equation is related to this chapter, the thing is half [the number of] the things minus the root of the remainder from the subtraction of the number from the product of the other half [the number of] the things. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{x=\left(\frac{1}{2}\sdot\frac{b}{a}\right)-\sqrt{\left(\frac{1}{2}\sdot\frac{b}{a}\right)^2-\frac{c}{a}}}}</math> | ||
| + | |style="text-align:right;"|ופעמים בחשבונות מה בבא אל ההשואה מזה הפרק הדבר יהיה מה{{#annot:term|845|5itx}}מחצית מ{{#annotend:5itx}}הדברים פחות שרש {{#annot:term|184,1236|nYQ2}}הנשאר מהוצאת{{#annotend:nYQ2}} המספר מכפל המחצית האחר מהדברים | ||
| + | |- | ||
| + | |Some calculations can be answered both ways, i.e. half [the number of] the things plus a root of the stated remainder, or half [the number of] the things minus a root of the stated remainder | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{x=\left(\frac{1}{2}\sdot\frac{b}{a}\right)\pm\sqrt{\left(\frac{1}{2}\sdot\frac{b}{a}\right)^2-\frac{c}{a}}}}</math> | ||
| + | |style="text-align:right;"|וחשבונות מה יוכל לענות בם בשני האופנים דהיינו מחצית הדברים ויותר שרש משארית האמור או מחצית הדברים פחות שרש השארית האמור | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:a+b=10, (a-b)²=20+¼|619|FlXs}}Divide ten into two parts such that when the difference between them is multiplied by itself the result is twenty and a quarter. | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle a+b=10\\\scriptstyle\left(a-b\right)^2=20+\frac{1}{4}\end{cases}</math> | ||
| + | |style="text-align:right;"|והנה המשל עשה לי מעשרה שני חלקים שכאשר הוכה ההבדל שבין זה לזה בעצמו יעשה עשרים ורביע{{#annotend:FlXs}} | ||
| + | |- | ||
| + | |Following the rule: | ||
| + | |style="text-align:right;"|תעשה כמו שאומר הכלל | ||
| + | |- | ||
| + | | | ||
| + | :defining: | ||
| + | :*one of the parts as a thing <math>\scriptstyle{\color{blue}{a=x}}</math> | ||
| + | |style="text-align:right;"|אנחנו צריכין להניח שאחד מהחלקים יהיה דבר אחד | ||
| + | |- | ||
| + | | | ||
| + | :*the other remains ten minus a thing <math>\scriptstyle{\color{blue}{b=10-x}}</math> | ||
| + | |style="text-align:right;"|והאחר ישאר עשרה פחות דבר אחד | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{x-\left(10-x\right)=2x-10}}</math> | ||
| + | |style="text-align:right;"|עתה תקח ההבדל שבין זה לזה שהוא שני דברים פחות עשרה | ||
| + | |- | ||
| + | | | ||
| + | ::::check: <math>\scriptstyle{\color{blue}{\left(10-x\right)+\left(2x-10\right)=x}}</math> | ||
| + | |style="text-align:right;"|ואם רצית לדעת כיצד יהיה ההבדל ב' דברים פחות עשרה<br> | ||
| + | תוסיף ב' דברים פחות עשרה על עשרה פחות דבר אחד שהוא החלק השני ויהיה לך דבר אחד<br> | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{a-b=x-\left(10-x\right)=2x-10}}</math> | ||
| + | |style="text-align:right;"|א"כ הנך רואה היטב כי להוציא מדבר אחד עשרה פחות דבר אחד ישאר ב' דברים פחות עשרה<br> | ||
| + | וכן הוא ההבדל מה שהחלק האחד גדול מהאחר | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{\left(2x-10\right)^2=100+4x^2-40x}}</math> | ||
| + | ::<math>\scriptstyle{\color{blue}{100+4x^2-40x=20+\frac{1}{4}}}</math> | ||
| + | |style="text-align:right;"|וזה ההבדל שהוא ב' דברי' פחות עשרה תכפול בעצמו ועולה ק' מספרים וד' צינסו פחות מ' דברי' שהם {{#annot:term|429,1247|a8Fj}}ישוו אל{{#annotend:a8Fj}} כ' ורביע | ||
| + | |- | ||
| + | | | ||
| + | :<span style=color:Green>'''Normalization''':</span> <math>\scriptstyle4x^2</math>: <math>\scriptstyle{\color{blue}{100+4x^2-40x=20+\frac{1}{4}\; /\div4}}</math> | ||
| + | |style="text-align:right;"|עתה צריכי' אנו לחלק כל ההשואה על כמות הצינסי דהיינו על ד' כפי הכלל האמור למעלה | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{x^2+25-10x=5+\frac{1}{16}}}</math> | ||
| + | |style="text-align:right;"|שעולה א' צינסו וכ"ה מספרים פחות עשרה דברים שוים לה' מספרים וחלק מי"ו | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{x^2+25-10x=5+\frac{1}{16}\; /-\left(5+\frac{1}{16}\right)}}</math> | ||
| + | |style="text-align:right;"|עתה צריכי' אנו להוציא המספרים הקטנים מכל אחד מהחלקים שהם ה' וחלק מי"ו | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{x^2+\left(19+\frac{15}{16}\right)-10x=0}}</math> | ||
| + | |style="text-align:right;"|וישאר לאחד מהחלקים לא כלום ולאחר ישאר י"ט וט"ו חלקים מי"ו | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{x^2+\left(19+\frac{15}{16}\right)-10x=0\; /+10x}}</math> | ||
| + | |style="text-align:right;"|ועתה תוסיף על כך אחד מהחלקים כל כך דברים כמו שאחד מהחלקים יש לו פחות | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{x^2+\left(19+\frac{15}{16}\right)+0=10x}}</math> | ||
| + | |style="text-align:right;"|ואחד מהם יהיה לו לא דבר ולחלק האחר יהיה לו עשרה דברים | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{x^2+\left(19+\frac{15}{16}\right)=10x}}</math> | ||
| + | |style="text-align:right;"|א"כ יהיה הנשאר לאחד מהחלקי' א' צינסו וי"ט מספרים וט"ו חלקים מי"ו שוה לעשרה דברים דהיינו לחלק האחר | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ועתה הנני מודיעך כי ההמשך שעשינו כאשר היה לנו ק' מספרי' וא' צינסו פחות מ' דברי' שוים אל כ' מספרים ורביע היה לו זה הפחיתות שההשואה לא הסכימה עם הכלל הנתון למעלה | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ו{{#annot:term|198|pIHj}}אמות זה{{#annotend:pIHj}} הוא כי לא {{#annot:term|181,2562|55eP}}הוצאו{{#annotend:55eP}} עדיין המספרי' הקטנים מכל אחד מהחלקי' גם לא {{#annot:term|178,1213|joz1}}נוספו ה{{#annotend:joz1}}דברים אע"פ שהחשבון יצא לפעל אמתי אנחנו {{#annot:term|784,1259|5Goa}}חלקנו על{{#annotend:5Goa}} כמות הצינסי קודם הזמן כמו שנראה בכלל הפרק האמור | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{100+4x^2-40x=20+\frac{1}{4}\; /-\left(20+\frac{1}{4}\right)}}</math> | ||
| + | |style="text-align:right;"|וראוי לנו להחזיק בזה האופן שאנחנו צריכי' להוציא המספר הקטן שהוא כ' ורביע מכל אחד מהחלקים | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(79+\frac{3}{4}\right)+4x^2-40x=0}}</math> | ||
| + | |style="text-align:right;"|וישאר לאחד מהחלקים לא שום מספר ואל האחר ע"ט וג' רביעי' וד' צינסי פחות מ' דברים | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(79+\frac{3}{4}\right)+4x^2-40x=0\; /+{\color{red}{40x}}}}</math> | ||
| + | |style="text-align:right;"|ואח"כ היה ראוי להוסיף מה שגורע מאחד מהחלקים לכל אחד מהחלקים | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{4x^2+\left(79+\frac{3}{4}\right)=40x}}</math> | ||
| + | |style="text-align:right;"|היה נשאר ולאחד מהחלקים נשאר ד' צינסו וע"ט וג' רביעי' ולחלק האחר כ' דברים | ||
| + | |- | ||
| + | | | ||
| + | :<math>\scriptstyle ax^2+c=bx</math> | ||
| + | |style="text-align:right;"|שהוא היטב מסכים לכלל הקודם האומ' הצינסי והמספרים שוים אל הדברים | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{x^2+\left(19+\frac{15}{16}\right)=10x}}</math> | ||
| + | |style="text-align:right;"|כי {{#annot:term|157,1223|CtRD}}בחלוק על{{#annotend:CtRD}} הצינסי עולה היטב כך כמו שעולה באופן האחר שהוא א' צינסו וי"ט מספרים וט"ו חלקים מי"ו שוים לי' דברים | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|עתה {{#annot:term|786,1369|KdWB}}נחצה ה{{#annotend:KdWB}}דברים בהמשכה אחר הכלל ויהיה לנו ה‫'<br> | ||
| + | ואלו הה' נכפלם בעצמם ועולה כ"ה<br> | ||
| + | ומהם תוציא המספר שהוא י"ט וט"ו חלקים מי"ו ויהיה לך שהדבר יהיה החצי האחד מהדברים שהוא ה' ויותר שרש ה' וחלק מי"ו<br> | ||
| + | וזה השרש הוא ב' ורביע<br> | ||
| + | א"כ יהיה שוה הדבר ז' ורביע אשר הנחת היותו ראשון החלקים | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle a=x&\scriptstyle=\left(\frac{1}{2}\sdot10\right)+\sqrt{\left(\frac{1}{2}\sdot10\right)^2-\left(19+\frac{15}{16}\right)}=5+\sqrt{5^2-\left(19+\frac{15}{16}\right)}\\&\scriptstyle=5+\sqrt{25-\left(19+\frac{15}{16}\right)}\\&\scriptstyle=5+\sqrt{5+\frac{1}{16}}\\&\scriptstyle=5+\left(2+\frac{1}{4}\right)=7+\frac{1}{4}\\\end{align}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{b=10-x=2+\frac{3}{4}}}</math> | ||
| + | |style="text-align:right;"|והחלק האחר הוא הנשאר עד עשרה שהוא ב' וג' רביעים | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|וזה החשבון נענה מן האופן הראשון שהוא מחצית הדברי' ויותר שרש הנשאר האמור | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ובאופן אחר אי אפשר לענות בו בהיות שהונח אל ההשואה האמורה למעלה בקחת ההבדל באופן האמור | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{\left(10-x\right)-x=10-2x}}</math> | ||
| + | |style="text-align:right;"|וסבת זה היא כי אם אמרת היות ההבדל באופן אחר שיוכל להיות רצוני עשרה פחות ב' דברים אפשר שיעלה החשבון כאמור דהיינו בפחות מה שהיה יכול להיות הדבר אם היה יכול להיות החלק הקטן | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|וזה הדבר אי אפשר לעשותו בהיות ההבדל ב' דברים פחות עשרה | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{2x>10}}</math> | ||
| + | |style="text-align:right;"|בעבור שב' דברים צריכי' להיות יותר מעשרה | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{x-\left(10-x\right)=2x-10}}</math> | ||
| + | |style="text-align:right;"|אם עשרה צריך להוציא מאותו שעולה ב' דברים | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{a=x=5+\sqrt{5+\frac{1}{16}}=7+\frac{1}{4}}}</math> | ||
| + | |style="text-align:right;"|והנה המשל אם הדבר יבא להיות ה' ושרש ה' וחלק מי"ו<br> | ||
| + | כי כאשר הושב אל מספר יבא להיות ז' ורביע כל החלק | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{2x=14+\frac{1}{2}}}</math> | ||
| + | |style="text-align:right;"|א"כ ב' דברים יבא להיות י"ד וחצי | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{2x-10=\left(14+\frac{1}{2}\right)-10=4+\frac{1}{2}}}</math> | ||
| + | |style="text-align:right;"|וההבדל נעשה ב' דברים פחות עשרה א"כ תוציא עשרה מי"ד וחצי וישאר ד' וחצי<br> | ||
| + | והנה ההבדל יבא להיות ד' וחצי | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(4+\frac{1}{2}\right)^2=20+\frac{1}{4}}}</math> | ||
| + | |style="text-align:right;"|וזאת ההכאה יעשה היטב כ' ורביע | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{a=x=5-\sqrt{5+\frac{1}{16}}=2+\frac{3}{4}}}</math> | ||
| + | |style="text-align:right;"|ואם תאמ' שהדבר יהיה ה' פחות שרש ה' וחלק מי"ו אשר כשהושב אל {{#annot:term|20,1268|HWds}}שלם{{#annotend:HWds}} יבא להיות ב' וג' רביעים | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{2x-10}}</math> impossible | ||
| + | |style="text-align:right;"|יהיה בלתי אפש' כי ב' דברים כאלה עשרה יוכל להוציא | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ולכן אי אפש' לענות בעשות ההבדל ב' דברים פחות עשרה כי אם מהיותר כפי מה שענינו | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|עוד בעבור הפרק החמישי | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:a+b=10, (b-a)²-a²=32|619|2N6x}}Divide ten into two parts such that when the smaller part is multiplied by itself and you subtract the product from the product of the difference between the parts by itself, 32 will remain. | ||
| + | :I ask: how much are the parts? | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle a+b=10\\\scriptstyle\left(b-a\right)^2-a^2=32\end{cases}</math> | ||
| + | |style="text-align:right;"|עשה לי מעשרה שני חלקים שכשהוכה החלק הקטן בעצמו ותוציא העולה מזה מהכאת ההבדל שיש בין החלקים בעצמו ישאר ל"ב<br> | ||
| + | אשאל כמה יבא להיות מהחלקים{{#annotend:2N6x}} | ||
| + | |- | ||
| + | |Following the rule: | ||
| + | |style="text-align:right;"|תעשה כמו שאומ' הכלל | ||
| + | |- | ||
| + | | | ||
| + | :defining: | ||
| + | :*the smaller part as a thing <math>\scriptstyle{\color{blue}{a=x}}</math> | ||
| + | |style="text-align:right;"|תניח שהחלק הקטון יהיה דבר אחד | ||
| + | |- | ||
| + | | | ||
| + | :*the other will be the remainder from ten which is ten minus a thing <math>\scriptstyle{\color{blue}{b=10-x}}</math> | ||
| + | |style="text-align:right;"|והאחר יבא להיות השארית עד עשרה שהוא עשרה פחות דבר אחד | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{x\sdot x=x^2}}</math> | ||
| + | |style="text-align:right;"|עתה תכה החלק הקטן שהוא דבר אחד בעצמו ויעלה א' צינסו | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{red}{\left(10-x\right)-x=10-2x}}</math> | ||
| + | |style="text-align:right;"|עתה תקח ההבדל אשר בין הקטן והגדול שהוא ב' פחות עשרה דברים | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{\left(10-2x\right)^2=4x^2+100-40x}}</math> | ||
| + | |style="text-align:right;"|ותכהו בעצמו ועלה ד' צינסו וק' מספרים פחות מ' דברים | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{\left(4x^2+100-40x\right)-x^2={\color{red}{3}}x^2+100-40x}}</math> | ||
| + | ::<math>\scriptstyle{\color{blue}{{\color{red}{3}}x^2+100-40x=32}}</math> | ||
| + | |style="text-align:right;"|עתה תוציא הכאת החלק הקטן שהוא א' צינסו מהכאת ההבדל בעצמו שעשינו שהיא ד' צינסי וק' מספרי' פחות מ' דברים וישאר עשרה צינסו וק' מספרים פחות מ' דברי' שוים אל ל"ב מספרים כפי השאלה הנתונה למעלה | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{{\color{red}{3}}x^2+100-40x=32\; /-32}}</math> | ||
| + | |style="text-align:right;"|עתה צריכים אנו להוציא המספר הקטון מכל אחד מן החלקים שהוא ל"ב | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{3x^2+68-40x=0}}</math> | ||
| + | |style="text-align:right;"|וישאר אל אחד מהחלקים אין מספר ולחלק האחר ג' צינסו וס"ח מספרים פחות מ' דברים | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{3x^2+68-40x=0\; /+40x}}</math> | ||
| + | |style="text-align:right;"|אח"כ תוסיף מ' דברים שהוא פוחת לאחד מהחלקים | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{3x^2+68=40x}}</math> | ||
| + | |style="text-align:right;"|ולכל חלק ישאר השואתו דהיינו לחלק האחד ג' צינסי וס"ח מספרים ולאחר מ' דברי‫'<br> | ||
| + | והנה יש לנו כי ג' צינסי וס"ח מספרי' הם שוים למ' דברי‫' | ||
| + | |- | ||
| + | | | ||
| + | :<span style=color:Green>'''Normalization''':</span> <math>\scriptstyle3x^2</math>: <math>\scriptstyle{\color{blue}{3x^2+68=40x\; /\div3}}</math> | ||
| + | |style="text-align:right;"|עתה צריכי' אנו לחלק כל ההשואה בכל כמות הצינסי שהם ג' כפי הכלל האמור למעלה | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{x^2+\left(22+\frac{2}{3}\right)=\left(13+\frac{1}{3}\right)x}}</math> | ||
| + | |style="text-align:right;"|ויגיע לנו א' צינסו וכ"ב מספרים וב' שלישי' שוים לי"ג דברים ושליש | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle a=x&\scriptstyle=\left[\frac{1}{2}\sdot\left(13+\frac{1}{3}\right)\right]-\sqrt{\left[\frac{1}{2}\sdot\left(13+\frac{1}{3}\right)\right]^2-\left(22+\frac{2}{3}\right)}\\&\scriptstyle=\left(6+\frac{2}{3}\right)-\sqrt{\left(6+\frac{2}{3}\right)^2-\left(22+\frac{2}{3}\right)}\\&\scriptstyle=\left(6+\frac{2}{3}\right)-\sqrt{\left(44+\frac{4}{9}\right)-\left(22+\frac{2}{3}\right)}\\&\scriptstyle=\left(6+\frac{2}{3}\right)-\sqrt{21+\frac{7}{9}}\\\end{align}}}</math> | ||
| + | |style="text-align:right;"|עתה ש{{#annot:term|970,2559|r7n6}}הושבה אל{{#annotend:r7n6}} א' צינסו תחלק הדברים לחצי שעולה ו' וב' שלישי'<br> | ||
| + | ותכם בעצמם ועולה מ"ד וד' תשיעיות<br> | ||
| + | תוציא מהם המספר שהוא כ"ב וב' שלישי' וישאר כ"א וז' תשיעיות<br> | ||
| + | ושרש אלו הכ"א וז' תשיעיות תוציא הנשאר ממחצית האחר מהדברים וככה ישוה הדבר שהוא החלק הראשון והקטן אשר הנחת<br> | ||
| + | א"כ יהיה לך מענה זה החשבון שהחלק הקטן הוא ו' וב' שלישית פחות שרש כ"א וז' תשיעיות | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|והושבה מן האופן השני שאמר הפרק החמישי | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{b=10-x=\left(3+\frac{1}{3}\right)+\sqrt{21+\frac{7}{9}}}}</math> | ||
| + | |style="text-align:right;"|והחלק האחר יבא להיות הנשאר עד עשרה שהוא ג' ושליש ושרש כ"א וז' תשיעיות | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{\left(10-x\right)-x=10-2x}}</math> | ||
| + | |style="text-align:right;"|ועתה הנני מודיעך שאתה אם לקחת ההבדל באופן האחד שאפש' לקחתו כפי מה שהראית קודם באופן הראשון שיבא להיות עשרה פחות ב' דברי' היה בא המענה כדומה לזה האמור למעלה | ||
| + | |- | ||
| + | | | ||
| + | ::the smaller part cannot be <math>\scriptstyle{\color{blue}{x}}</math> | ||
| + | |style="text-align:right;"|ובאופן אחר אי אפש' לענותה בהניח שהחלק הקטן הוא דבר אחד בבא החשבון אל זה הראש | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|א"כ החשבון אי אפשר להגיע תשובתו כי אם מספר פחות שרש | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{a^2<\left(b-a\right)^2}}</math> | ||
| + | |style="text-align:right;"|מפני שהחלק הקטן מוכה בעצמו צריך שיעשה פחות מההבדל מוכה בעצמו | ||
| + | |- | ||
| + | | | ||
| + | ::since <math>\scriptstyle{\color{blue}{\left(b-a\right)^2-a^2=32}}</math> | ||
| + | |style="text-align:right;"|אחר שבהוצאת הכאת הקטן מן הכאת ההבדל צריך שישאר ל"ב | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ומפני זה החשבון האמור לא יתכן לשומו בזה הפרק כי אם בשני האופנים האמורים למעלה | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(6+\frac{1}{3}\right)+\sqrt{21+\frac{7}{9}}}}</math> greater than the sum of the two parts, which should be ten | ||
| + | |style="text-align:right;"|ומפני כי באמרנו היות הדבר מספר ושרש היה נראה שהחלק הקטן שהוא ששה וב' שלישי' ושרש מכ"א וז' תשיעיות יהיה יותר משני החלקים שהם ראויים להיות עשרה | ||
| + | |- | ||
| + | | | ||
| + | :the part cannot be greater than the whole | ||
| + | |style="text-align:right;"|וזה דבר נמנע שהחלק יהיה גדול מהכל | ||
| + | |- | ||
| + | | | ||
| + | :Check: | ||
| + | |style="text-align:right;"|ואם רצית לראות ראית זה מבוארת | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{a=\left(6+\frac{2}{3}\right)-\sqrt{21+\frac{7}{9}}=\left(6+\frac{2}{3}\right)-\left(4+\frac{2}{3}\right)=2}}</math> | ||
| + | |style="text-align:right;"|תקח השרש מכ"א וז' תשיעיות שהוא ד' וב' שלישי‫'<br> | ||
| + | ותוציאם מו' וב' שלישי' ישאר ב‫'<br> | ||
| + | וככה יבא להיות החלק הקטן | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{a=10-2=8}}</math> | ||
| + | |style="text-align:right;"|והחלק הגדול יבא להיות הנשאר עד עשרה שהוא ח‫' | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{b-a=8-2=6}}</math> | ||
| + | |style="text-align:right;"|וההבדל יהיה ו' כשתפיל ב' מח‫' | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(b-a\right)^2=6^2=36}}</math> | ||
| + | |style="text-align:right;"|תכה ו' בעצמו ועושה ל"ו | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{a^2=2^2=4}}</math> | ||
| + | |style="text-align:right;"|עתה תכה החלק הקטן שהוא ב' בעצמו ועושה ד‫' | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{\left(b-a\right)^2-a^2=36-4=32}}</math> | ||
| + | |style="text-align:right;"|תוציאם מל"ו וישאר ל"ב כפי השאלה האמורה למעלה | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|עוד בעבור הפרק האמור למעלה | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:a+b=10, ab=21|619|Ibmi}}Divide ten into two parts such that when one [part] is multiplied by the other the result is 21. | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle a+b=10\\\scriptstyle a\sdot b=21\end{cases}</math> | ||
| + | |style="text-align:right;"|תעשה לי מעשרה שני חלקים שכשהוכה האחד באחר יעשה כ"א{{#annotend:Ibmi}} | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{a=x}}</math> | ||
| + | |style="text-align:right;"|זהו כלל נהוג תניח שאחד מהחלקים יהיה דבר אחד | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{b=10-x}}</math> | ||
| + | |style="text-align:right;"|והאחר ישאר עשרה פחות דבר אחד | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{x\sdot\left(10-x\right)=10x-x^2}}</math> | ||
| + | |style="text-align:right;"|תכה דבר אחד בעשרה פחות דבר אחד ועולה עשרה דברים פחות א' צינסו | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{10x-x^2=21}}</math> | ||
| + | |style="text-align:right;"|ואלו העשרה דברים פחות א' צינסו הם שוים אל כ"א | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{10x-x^2=21\; /+x^2}}</math> | ||
| + | |style="text-align:right;"|עתה צריך אתה לתת א' צינסו לכל אחד מהחלקים | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{10x=x^2+21}}</math> | ||
| + | |style="text-align:right;"|ויהיה לך עשרה דברים שוים לא' צינסו וכ"א מספרים | ||
| + | |- | ||
| + | | | ||
| + | :Halve [the number of] the things; the result is 5. | ||
| + | |style="text-align:right;"|עתה תחצה הדברים ויגיע ה‫' | ||
| + | |- | ||
| + | | | ||
| + | :Multiply 5 by itself; it yields 25. | ||
| + | |style="text-align:right;"|תכה ה' בעצמו ועושה כ"ה | ||
| + | |- | ||
| + | | | ||
| + | :Now, subtract the number, which is 21, from 25; 4 remains. | ||
| + | |style="text-align:right;"|עתה תוציא המספר שהוא כ"א מכ"ה וישאר ד‫' | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ושרש אלו הד' תחבר מהחלק האחר שהוא ממחצית הדברים שהוא ה' ויהיה לך ה' ושרש ד‫' | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|או אם תרצה אמור ה' פחות שרש ד‫'<br> | ||
| + | וכן ישוה הדבר | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle x&\scriptstyle=\left(\frac{1}{2}\sdot10\right)\pm\sqrt{\left(\frac{1}{2}\sdot10\right)^2-21}=5\pm\sqrt{5^2-21}\\&\scriptstyle=5\pm\sqrt{25-21}\\&\scriptstyle=5\pm\sqrt{4}\\\end{align}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|והנשאר עד עשרה הוא החלק האחר | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|א"כ הנך יכול לענות ביותר ובפחות מה ששוה הדבר | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|עתה ראית כל התשובות אשר אפש' לעשותם בעבור הפרק החמישי האמור | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|עוד בעבור הפרק החמישי האמור | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:a+b=10, 3a=b√8|619|kTPd}}Divide ten into two parts such that when one [part] is multiplied by 3 the product is the same as the product of the other by the root of 8. | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle a+b=10\\\scriptstyle3a=b\sdot\sqrt{8}\end{cases}</math> | ||
| + | |style="text-align:right;"|עשה לי מעשרה ב' חלקים אשר כשהוכה האחד בג' יעשה כך כמו שעולה הכאת האחר בשרש ח‫'{{#annotend:kTPd}} | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{a=x}}</math> | ||
| + | |style="text-align:right;"|תניח שאחד מהחלקים יהיה דבר אחד | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{b=10-x}}</math> | ||
| + | |style="text-align:right;"|והאחר מחוייב שיהיה עשרה פחות דבר אחד | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{3\sdot x=3x}}</math> | ||
| + | |style="text-align:right;"|עתה תכה ג' בדבר אחד ועושה ג' דברים | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle\sqrt{8}\sdot\left(10-x\right)&\scriptstyle=\sqrt{8}\sdot\sqrt{\left(10-x\right)^2}\\&\scriptstyle=\sqrt{8}\sdot\sqrt{100-20x+x^2}\\&\scriptstyle=\sqrt{800-160x+8x^2}\\\end{align}}}</math> | ||
| + | ::[error]: <math>\scriptstyle{\color{blue}{800-160x+8x^2=3x}}</math> | ||
| + | |style="text-align:right;"|ותכה שרש ח' בעשרה פחות דבר אחד<br> | ||
| + | שעושה ק' פחות כ' דברים ויותר א' צינסו<br> | ||
| + | דהיינו בהביא עשרה פחות דבר אחד אל השרש<br> | ||
| + | עתה תכפול ח' בשרש ק' פחות כ' דברים ויותר א' צינסו שעושה ת"ת פחות ק"ס דברים ויותר ח' צינסו<br> | ||
| + | שהם שוים לג' דברים | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{800-160x+8x^2=3x\; /+160x}}</math> | ||
| + | |style="text-align:right;"|עתה תשוה החלקים ותן לכל חלק ק"ס דברים | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{163x=800+8x^2}}</math> | ||
| + | |style="text-align:right;"|ויהיה לך קס"ג דברים להיות שוה לת"ת מספרי' וח' צינסי | ||
| + | |- | ||
| + | | | ||
| + | :<span style=color:Green>'''Normalization''':</span> <math>\scriptstyle8x^2</math>: <math>\scriptstyle{\color{blue}{163x=800+8x^2\; /\div8}}</math> | ||
| + | |style="text-align:right;"|תחלק א"כ כל דבר על הצינסי להשיב כל דבר אל א' צינסי | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{x^2+\left(20+\frac{3}{8}\right)x=100}}</math> | ||
| + | |style="text-align:right;"|שיגיע מזה א' צינסו וכ' דברים וג' שמיניות שהוא ק' מספרים | ||
| + | |- | ||
| + | | | ||
| + | :Now, halve [the number of] the things; it is ten and 3 parts of 16. | ||
| + | |style="text-align:right;"|עתה תחצה הדברים שהם עשרה וג' חלקים מי"ו | ||
| + | |- | ||
| + | | | ||
| + | :Multiply it by itself; it yields 103 and 201 parts of 256. | ||
| + | |style="text-align:right;"|תכפלם בעצמם שעושה ק"ג ור"א חלקים מרנ"ו | ||
| + | |- | ||
| + | | | ||
| + | :Subtract from it the number, which is 100; 3 and 201 parts of 256 remain. | ||
| + | |style="text-align:right;"|{{#annot:term|181,1251|2fXN}}תפיל מהם{{#annotend:2fXN}} המספר שהוא ק' ישאר ג' ור"א חלקים מרנ"ו | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ושרש זה הג' ור"א חלקים מרנ"ו ויותר מחצית הדברים שוה הדבר | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle x&\scriptstyle=\left[\frac{1}{2}\sdot\left(20+\frac{3}{8}\right)\right]+\sqrt{\left[\frac{1}{2}\sdot\left(20+\frac{3}{8}\right)\right]^2-100}=\left(10+\frac{3}{16}\right)+\sqrt{\left(10+\frac{3}{16}\right)^2-100}\\&\scriptstyle=\left(10+\frac{3}{16}\right)+\sqrt{\left(103+\frac{201}{256}\right)-100}\\&\scriptstyle=\left(10+\frac{3}{16}\right)+\sqrt{3+\frac{201}{256}}\\\end{align}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ואתה הנחת שאחד מהחלקי' היה דבר אחד א"כ היה שרש ג' ור"א חלקים מרנ"ו יותר ממחצית הדברים | ||
| + | |- | ||
| + | | | ||
| + | ::[error]: <math>\scriptstyle{\color{blue}{b=10-x=\sqrt{3+\frac{201}{256}}-\left(10+\frac{3}{16}\right)}}</math> | ||
| + | |style="text-align:right;"|והחלק האחר היה הנשאר עד עשרה שהוא שרש ג' ור"א חלקים מרנ"ו פחות מן עשרה וג' חלקים מן י"ו | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|אני האמנתי שזה היה נעשה היטב ועתה בעשות הנסיון מצאתיהו משובש ועתה אעשנו כראוי להיות ואפש' להוליכו מן הפרק החמישי | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{a=x}}</math> | ||
| + | |style="text-align:right;"|והחלק הראשון אניח שהיה דבר אחד | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{b=10-x}}</math> | ||
| + | |style="text-align:right;"|והשני צריך שיהיה עשרה פחות דבר אחד | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ואזכירך שצריך להשיב כל דבר אל שרש | ||
| + | |- | ||
| + | | | ||
| + | :::converting 3 into a root: <math>\scriptstyle{\color{blue}{3=\sqrt{9}}}</math> | ||
| + | |style="text-align:right;"|עתה תשיב ג' אל שרש ועושה ט‫' | ||
| + | |- | ||
| + | | | ||
| + | :::converting x into a root: <math>\scriptstyle{\color{blue}{x=\sqrt{x^2}}}</math> | ||
| + | |style="text-align:right;"|וכן תשיב דבר אחד אל שרש ועושה א' צינסו | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{3x=\sqrt{9x^2}}}</math> | ||
| + | |style="text-align:right;"|אח"כ תכפול ט' בא' צינסו ועושה ט' צינסי וזה לך בעד אחד מהחלקים | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{10-x=\sqrt{100-20x+x^2}}}</math> | ||
| + | |style="text-align:right;"|אח"כ כמו שהשבותה למעלה השב עשרה פחות דבר אחד אל שרש ועושה שרש ק' פחות כ' דברים ויותר א' צינסו | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|וזה תכפול בשרש ח' ועושה שורש ת"ת פחות ק"ס דברים ויותר ח' צינסי אשר הם שוים אל ט' צינסי | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{\left(10-x\right)\sdot\sqrt{8}=\sqrt{100-20x+x^2}\sdot\sqrt{8}=\sqrt{800-160x+8x^2}=\sqrt{9x^2}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{800-160x+8x^2=9x^2\quad/+160x}}</math> | ||
| + | |style="text-align:right;"|ועתה תשוה החלקי' ותן לכל אחד ק"ס דברי‫' | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{9x^2+160x=800+8x^2}}</math> | ||
| + | |style="text-align:right;"|ויהיה לך ט' צינסי וק"ס דברים שוים לת"ת מספרי' וח' צינסי | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{9x^2-8x^2}}</math> | ||
| + | |style="text-align:right;"|תשוה עוד והוצא ח' צינסי מט' צינסי | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{x^2+160x=800}}</math> | ||
| + | |style="text-align:right;"|ויהיה לך א' צינסו וק"ס דברי' שוים אל ת"ת מספרי‫' | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|חלק עתה על הצינסי ויגיע לך ק"ס דברי' ות"ת מספרי‫' | ||
| + | |- | ||
| + | | | ||
| + | :Halve [the number of] the things; you have 80. | ||
| + | |style="text-align:right;"|תחצה הדברים ויהיה לך פ‫' | ||
| + | |- | ||
| + | | | ||
| + | :Multiply it by itself; it yields 6400. | ||
| + | |style="text-align:right;"|תכפלם בעצמם ועושה ו' אלפים ות‫' | ||
| + | |- | ||
| + | | | ||
| + | :Add it to the number, which is 800; it yields 7200. | ||
| + | |style="text-align:right;"|תחברם אל המספר שהוא ת"ת ויעשה ז' אלפים ור‫' | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ושרש ז' אלפים ור' פחות מחצית הדברים שהוא פ' היה אחד מהחלקי' שהוכה בשרש ט‫' | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle a=x&\scriptstyle=\sqrt{\left(\frac{1}{2}\sdot160\right)^2+800}-\left(\frac{1}{2}\sdot160\right)=\sqrt{80^2+800}-80\\&\scriptstyle=\sqrt{6400+800}-80\\&\scriptstyle=\sqrt{7200}-80\\\end{align}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|והחלק האחר היה הנשאר עד עשרה אשר הוכפל בשרש ח' וזה החלק יגיע להיות צ' פחות שרש ז' אלפים ור‫' | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ונעשה בעבור הפרק החמישי | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|עוד בעבור הפרק החמישי החשבון האמור רצוני להניחו אל הפרק החמישי | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{b=x}}</math> | ||
| + | |style="text-align:right;"|ותניח כי היה אחד מהחלקים דבר אחד | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{a=10-x}}</math> | ||
| + | |style="text-align:right;"|והאחר היה עשרה פחות דבר אחד | ||
| + | |- | ||
| + | | | ||
| + | ::converting all the parts into roots: | ||
| + | |style="text-align:right;"|תשיב כל החלקים אל שרש | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{x=\sqrt{x^2}}}</math> | ||
| + | |style="text-align:right;"|ויהיה לך א' צינסו לחלק אחד | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{10-x=\sqrt{100-20x+x^2}}}</math> | ||
| + | |style="text-align:right;"|וק' מספרים פחות כ' דברים וא' צינסו לחלק האחר | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{x\sdot\sqrt{8}=\sqrt{x^2\sdot8}=\sqrt{8x^2}}}</math> | ||
| + | |style="text-align:right;"|ועתה תכפול החלק הראשון שהוא א' צינסו בח' ועושה ח' צינסי וזהו לך לחלק אחד | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|תכפול השני בט' ויהיה לך תת"ק מספרי' פחות ק"פ דברים ויותר ט' צינסו שהם שוים לח' צינסי | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{3\sdot\left(10-x\right)=\sqrt{9\sdot\left(100-20x+x^2\right)}=\sqrt{900-180x+9x^2}}}</math> | ||
| + | :<math>\scriptstyle{\color{blue}{900-180x+9x^2=8x^2}}</math> | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{900-180x+9x^2=8x^2\quad/+180x}}</math> | ||
| + | |style="text-align:right;"|תשוה החלקים ותן ק"פ דברים לכל אחד מן החלקים | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{8x^2+180x=900+9x^2}}</math> | ||
| + | |style="text-align:right;"|ויהיה לך ח' צינסי וק"פ דברים שוים אל תת"ק מספרים וט' צינסי | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{9x^2-8x^2}}</math> | ||
| + | |style="text-align:right;"|{{#annot:term|865,1246|5GnA}}השוה{{#annotend:5GnA}} עוד החלקי' והוציא ח' צינסי מט' צינסי | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{180x=900+x^2}}</math> | ||
| + | |style="text-align:right;"|ויהיה לך ק"פ דברים שוים לתת"ק מספרי' וא' צינסו | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|תחלק על הצינסו | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{180x=900+x^2}}</math> | ||
| + | |style="text-align:right;"|ויהיה לך ק"פ דברים שוים אל תת"ק מספרים וא' צינסו | ||
| + | |- | ||
| + | | | ||
| + | :Halve [the number of] the things; you have 90. | ||
| + | |style="text-align:right;"|תחצה הדברים ויהיה לך צ‫' | ||
| + | |- | ||
| + | | | ||
| + | :Multiply it by itself; you have 8100. | ||
| + | |style="text-align:right;"|תכם בעצמם ויהיה לך ח' אלפים וק‫' | ||
| + | |- | ||
| + | | | ||
| + | :Subtract the number; you are left with 7200. | ||
| + | |style="text-align:right;"|תוציא המספרים וישאר לך ז' אלפים ור‫' | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ומשרש זה תוציא מחצית הדברי' וככה ישוה הדבר | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{x=\left(\frac{1}{2}\sdot180\right)-\sqrt{\left(\frac{1}{2}\sdot180\right)^2-900}=90-\sqrt{90^2-900}=90-\sqrt{8100-900}=90-\sqrt{7200}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ואתה הנחת שהחלק הראשון היה דבר אחד א"כ היה צ' פחות שרש ז' אלפים ור‫' | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{b=10-x=\sqrt{7200}-80}}</math> | ||
| + | |style="text-align:right;"|והחלק האחר היה הנשאר עד עשרה שהוא שרש ז' אלפים ור' פחות פ‫' | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ונעשה עם הפרק החמישי | ||
| + | |- | ||
| + | |<math>\scriptstyle\begin{cases}\scriptstyle a+b=16\\\scriptstyle a\sdot b=48\end{cases}</math> | ||
| + | |style="text-align:right;"|עוד בעבור הפרק האמור עשה לי מי"ו שני חלקים שכאשר הוכה האחד באחר יעשה מ"ח | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{a=x}}</math> | ||
| + | |style="text-align:right;"|תניח שאחד החלקים היה א' דבר | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{b=16-x}}</math> | ||
| + | |style="text-align:right;"|והיה האחר בהכרח י"ו פחות דבר אחד | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{x\sdot\left(16-x\right)=16x-x^2}}</math> | ||
| + | ::<math>\scriptstyle{\color{blue}{16x-x^2=48}}</math> | ||
| + | |style="text-align:right;"|עתה תכה דבר אחד בי"ו פחות דבר אחד ועושה י"ו דברים פחות א' צינסו<br> | ||
| + | שהם שוים אל מ"ח | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{16x=48+x^2}}</math> | ||
| + | |style="text-align:right;"|תשוה א"כ החלקי' ויהיה לך י"ו דברים שוים למ"ח מספרי' וא' צינסו | ||
| + | |- | ||
| + | | | ||
| + | :Halve [the number of] the things; you have 8 things. | ||
| + | |style="text-align:right;"|תחצה הדברי' ויהיה לך ח' דברים | ||
| + | |- | ||
| + | | | ||
| + | :Multiply it by itself; it yields 64. | ||
| + | |style="text-align:right;"|תכם בעצמם ויעשה ס"ד | ||
| + | |- | ||
| + | | | ||
| + | :Subtract from it the number, which is 48; 16 remains. | ||
| + | |style="text-align:right;"|הוצא מזה המספר שהוא מ"ח ישאר י"ו | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ותוציא שרש הי"ו ממחצית הדברים ויהיה לך לחלק האחד ח' פחות שרש י"ו | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|או אם תרצה אמור השרש מי"ו תחבר על מחצית הדברים וככה ישוה הדבר | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{x=\left(\frac{1}{2}\sdot16\right)\pm\sqrt{\left(\frac{1}{2}\sdot16\right)^2-48}=8\pm\sqrt{8^2-48}=8\pm\sqrt{64-48}=8\pm\sqrt{16}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ואתה הנחת דבר אחד | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{x_1=8-\sqrt{16}=8-4=4}}</math> | ||
| + | |style="text-align:right;"|א"כ היה ח' פחות שרש י"ו שהוא ד' וישאר ד‫' | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{x_2=8+\sqrt{16}=8+4=12}}</math> | ||
| + | |style="text-align:right;"|או אם תרצה אמור היה שרש י"ו שהוא ד' ויותר ח' שיבא להיות י"ב | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|וכן יגיע באופן האחד כמו באחר כי אם תשים החלק הראשון י"ב האחר יהיה ד‫' | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|וכן הוא טבע זה הפרק החמשי | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== Chapter Six ==== | ||
| + | |||
| + | |style="width:45%;text-align:right;"|<big>הפרק הששי</big> | ||
| + | |- | ||
| + | |Its nature is when things and numbers are equal to squares. | ||
| + | :<math>\scriptstyle bx+c=ax^2</math> | ||
| + | |style="text-align:right;"|וטבעו כאשר הדברים והמספרים יהיו שוים אל הצינסי | ||
| + | |- | ||
| + | |The whole equation should be divided by the number of the squares. | ||
| + | |style="text-align:right;"|צריך לחלק כל ההשואה על כמות הצינסי | ||
| + | |- | ||
| + | |Then, the things should be divided into halves. | ||
| + | |style="text-align:right;"|ואח"כ לחלק הדברים לחצאים | ||
| + | |- | ||
| + | |One of the halves is multiplied by itself | ||
| + | |style="text-align:right;"|ולכפול אחד מהחצאים בעצמו | ||
| + | |- | ||
| + | |Add this product to the number. | ||
| + | |style="text-align:right;"|ואותה ההכאה תחבר על המספרים | ||
| + | |- | ||
| + | |The root of this sum plus half [the number of] the things is equal to the thing. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{x=\sqrt{\left(\frac{\frac{b}{a}}{2}\right)^2+\frac{c}{a}}+\frac{\frac{b}{a}}{2}}}</math> | ||
| + | |style="text-align:right;"|ושרש אותו הסך ויותר המחצית האחד מהדברים יהיה שוה הדבר | ||
| + | |- | ||
| + | | | ||
| + | *Find me a number such that when multiplied by ten and the product is added to 12 the result is the same as that number when multiplied by itself. | ||
| + | :<math>\scriptstyle10a+12=a^2</math> | ||
| + | |style="text-align:right;"|המשל תמצא לי מספר אחד שכאשר הוכה בעשרה ואותה ההכאה תחובר אל י"ב יעשה כמו המספר ההוא מוכה בעצמו | ||
| + | |- | ||
| + | | | ||
| + | :Proceed as its rule requires: | ||
| + | |style="text-align:right;"|תעשה כמו שרוצה זה הכלל שלו | ||
| + | |- | ||
| + | | | ||
| + | :Suppose the number is one thing [<math>\scriptstyle{\color{blue}{a=x}}</math>]. | ||
| + | |style="text-align:right;"|תניח שהמספר הוא דבר אחד | ||
| + | |- | ||
| + | | | ||
| + | :When it is multiplied by ten, the result is ten things. | ||
| + | :<math>\scriptstyle{\color{blue}{10\sdot x=10x}}</math> | ||
| + | |style="text-align:right;"|וכאשר הוכה בעשרה יעלה עשרה דברים | ||
| + | |- | ||
| + | | | ||
| + | :Now, add 12 to this product; you have ten things and 12 numbers that should be equal to one square, i.e. one thing multiplied by itself. | ||
| + | :<math>\scriptstyle{\color{blue}{10x+12=x^2}}</math> | ||
| + | |style="text-align:right;"|עתה תחבר אל זאת ההכאה י"ב ויהיה לך עשרה דברי' וי"ב מספרים אשר צריך שיהיו שוים אל א' צינסו דהיינו דבר אחד שהיה המספר מוכה בעצמו | ||
| + | |- | ||
| + | | | ||
| + | :Pursuing the above rule: | ||
| + | |style="text-align:right;"|עתה תרדוף כפי הכלל האמור למעלה | ||
| + | |- | ||
| + | | | ||
| + | :One square equals ten things plus 12 numbers. | ||
| + | :<math>\scriptstyle{\color{blue}{x^2=10x+12}}</math> | ||
| + | |style="text-align:right;"|שיבא א' צינסו שוה לעשרה דברים וי"ב מספרי‫' | ||
| + | |- | ||
| + | | | ||
| + | :Now, halve the number of the things; the result is 5. | ||
| + | |style="text-align:right;"|עתה תחצה המספרי' הדברים ויגיע ה‫' | ||
| + | |- | ||
| + | | | ||
| + | :Multiply it by itself; it is 25. | ||
| + | |style="text-align:right;"|תכם בעצמם ויהיה כ"ה | ||
| + | |- | ||
| + | | | ||
| + | :Add this product to 12; you get 37. | ||
| + | |style="text-align:right;"|וזאת ההכאה תחבר על י"ב ויהיה לך ל"ז | ||
| + | |- | ||
| + | | | ||
| + | :The root of 37 plus the other half of [the number of] the things is the thing, i.e. the number. | ||
| + | |style="text-align:right;"|ושר' ל"ז ושורש המחצית האחר מהדברי' יבא להיות הדבר דהיינו המספר | ||
| + | |- | ||
| + | | | ||
| + | :Hence, you receive that the number is 5 plus a root of 37. | ||
| + | |style="text-align:right;"|א"כ יהיה לך שהמספר יהיה ה' ושרש ל"ז | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{a=x=\left(\frac{1}{2}\sdot10\right)+\sqrt{\left(\frac{1}{2}\sdot10\right)^2+12}=5+\sqrt{5^2+12}=5+\sqrt{25+12}=5+\sqrt{37}}}</math> | ||
| + | |- | ||
| + | |It is solved according to chapter six. | ||
| + | |style="text-align:right;"|ונעשה בעבור הפרק הששי | ||
| + | |- | ||
| + | |Another calculation given for the said chapter: | ||
| + | |style="text-align:right;"|עוד זה החשבון יושם בעבור הפרק האמור | ||
| + | |- | ||
| + | | | ||
| + | *Find me a number such that when we subtract from it its third and its quarter and the remainder is multiplied by itself the result is that number plus one. | ||
| + | :<math>\scriptstyle\left(a-\frac{1}{3}a-\frac{1}{4}\right)^2=a+1</math> | ||
| + | |style="text-align:right;"|תמצא לי מספר אחד שכאשר {{#annot:term|181,1251|1Vvm}}נפיל ממנו{{#annotend:1Vvm}} שלישיתו ורביעיתו ומה שישאר יכפל בעצמו יעשה המספר ואחד יותר | ||
| + | |- | ||
| + | | | ||
| + | :<span style=color:Green>'''Normalization''':</span> Now, divide by [the number of] the squares and you get one square - it is as if you say: divide 25 parts of 144 of a square by 25 parts of 144; you get 1, which is one square. | ||
| + | :<math>\scriptstyle{\color{blue}{\frac{25}{144}x^2\div\frac{25}{144}=x^2}}</math> | ||
| + | |style="text-align:right;"|עתה תחלק על הצינסי ויהיה לך א' צינסו<br> | ||
| + | וזהו כאלו תאמר תחלק כ"ה חלקים מקמ"ד בצינסו על כ"ה חלקים מקמ"ד ויגיע לך א' שהוא א' צינסו | ||
| + | |- | ||
| + | | | ||
| + | :Divide one thing by 25 parts of 144; you get 5 and 19 parts of 25 of a drama. | ||
| + | :<math>\scriptstyle{\color{blue}{{\color{red}{1}}\div\frac{25}{144}=5+\frac{19}{25}}}</math> | ||
| + | |style="text-align:right;"|עתה תחלק דבר אחד על כ"ה חלקים מקמ"ד ויגיע לך ה' וי"ט חלקי' מכ"ה בדראמ‫' | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle\left(5+\frac{19}{25}\right)x+\left(5+\frac{19}{25}\right)=x^2</math> | ||
| + | |style="text-align:right;"|וכן יהיה לך ה' דברים וי"ט חלקי' מכ"ה בדבר וה' דראמ' וי"ט חלקים מכ"ה בדרמ' {{#annot:term|865,1246|7zzS}}תשוה ל{{#annotend:7zzS}}א' צינסו | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|תחצה הדברים שהם ב' וכ"ב חלקי' מכ"ה<br> | ||
| + | ותכם בעצמם ועושים ח' וקפ"ד חלקים מתרכ"ה<br> | ||
| + | וזה תחבר עם המספר שהוא ה' וי"ט חלקים מכ"ה ויגיע לך לסך י"ד ול"ד חלקים מתרכ"ה<br> | ||
| + | ושרש י"ד ול"ד חלקי' מתרכ"ה {{#annot:term|178,1215|C3R4}}בהתחברו עם{{#annotend:C3R4}} מחצית הדברים שהוא ב' וכ"ב חלקי' מכ"ה ככה שוה הדבר<br> | ||
| + | ואתה הנחת שהמספר היה דבר אחד א"כ היה ב' וכ"ב חלקי' מכ"ה ושרש י"ד ול"ד חלקים מתרכ"ה | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle a=x&\scriptstyle=\left[\frac{1}{2}\sdot\left(5+\frac{19}{25}\right)\right]+\sqrt{\left[\frac{1}{2}\sdot\left(5+\frac{19}{25}\right)\right]^2+\left(5+\frac{19}{25}\right)}=\left(2+\frac{22}{25}\right)+\sqrt{\left(2+\frac{22}{25}\right)^2+\left(5+\frac{19}{25}\right)}\\&\scriptstyle=\left(2+\frac{22}{25}\right)+\sqrt{\left(8+\frac{184}{625}\right)+\left(5+\frac{19}{25}\right)}=\left(2+\frac{22}{25}\right)+\sqrt{14+\frac{34}{625}}\\\end{align}}}</math> | ||
| + | |- | ||
| + | |Solved according to [chapter] six. | ||
| + | |style="text-align:right;"|וכך היה ונעשה בעד הששי | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | === <span style=color:Green>Complex Equations</span> === | ||
| + | |||
| + | | | ||
| + | |- | ||
| + | |Know that here begin other chapters that are derived from the six chapters written above, in which squares, cubes, and squares of squares are specified, as you will see further in this book explained well. | ||
| + | |style="width:45%; text-align:right;"|דע כי בכאן מתחיל פרקים אחרים אשר הם מוצאים מן הו' פרקים הכתובים למעלה ובהם יהיו נקובים צינסי ומעוקבים וצינסי מצינסי כמו שתראה בהמשך זה הספר מבואר היטב | ||
| + | |- | ||
| + | | | ||
| + | ==== Chapter Seven ==== | ||
| + | |||
| + | |style="text-align:right;"|<big>הפרק השביעי</big> | ||
| + | |- | ||
| + | |When cubes are equal to numbers: | ||
| + | :<math>\scriptstyle ax^3=c</math> | ||
| + | |style="text-align:right;"|כאשר המעוקבים יהיו שוים אל המספרי‫' | ||
| + | |- | ||
| + | |The numbers should be divided by the cubes | ||
| + | |style="text-align:right;"|צריך לחלק המספרים על המעוקבי‫' | ||
| + | |- | ||
| + | |The cube root of the result is equal to the thing. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{x=\sqrt[3]{\frac{c}{a}}}}</math> | ||
| + | |style="text-align:right;"|והעולה מזה הנה שרשו המעוקב יבא לשוות הדבר | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:a/b=¾;a²b=288|618|7M0L}}Find me two numbers such that the one is a part of the other as 3 is of 4; | ||
| + | :and when the smaller is multiplied by itself, and the product is multiplied by the larger, it yields 288. | ||
| + | :I ask: how much will each number be? | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle a:b=3:4\\\scriptstyle a^2\sdot b=288\end{cases}</math> | ||
| + | |style="text-align:right;"|תמצא לי שני מספרים שיהיה האחד חלק מהאחר כמו שהוא ג' מד‫'<br> | ||
| + | וכשיוכה הקטן בעצמו ו{{#annot:term|876,1233|QU3I}}היוצא{{#annotend:QU3I}} תכה בגדול יעשה רפ"ח<br> | ||
| + | אשאל כמה כל אחד מהמספרי‫'{{#annotend:7M0L}} | ||
| + | |- | ||
| + | | | ||
| + | :This is its rule: | ||
| + | |style="text-align:right;"|זהו הכלל שלו | ||
| + | |- | ||
| + | | | ||
| + | :Suppose one of the numbers is three things [<math>\scriptstyle{\color{blue}{a=3x}}</math>] and the other is four things [<math>\scriptstyle{\color{blue}{b=4x}}</math>] | ||
| + | |style="text-align:right;"|תניח אחד מהמספרים יהיה ג' דברים והאחר יהיה ד' דברים | ||
| + | |- | ||
| + | | | ||
| + | :Multiply the smaller, which is 3, by itself; the result is 9 squares. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(3x\right)^2=9x^2}}</math> | ||
| + | |style="text-align:right;"|תכה הקטון שהוא ג' בעצמו ועולה ט' צינסי | ||
| + | |- | ||
| + | | | ||
| + | :Then, multiply 9 squares by the greater number, which is 4 things; the result is 36 cubes that are equal to 288. | ||
| + | :<math>\scriptstyle{\color{blue}{9x^2\sdot4x=36x^3=288}}</math> | ||
| + | |style="text-align:right;"|ואח"כ תכה ט' צינסי במספר הגדול שהוא ד' דברים ועולה ל"ו מעוקבים שהם שוים אל רפ"ח | ||
| + | |- | ||
| + | | | ||
| + | :Pursue the above said rule: | ||
| + | |style="text-align:right;"|עתה תרדוף כפי הכלל האמור | ||
| + | |- | ||
| + | | | ||
| + | :Divide 288 by 36; the result is 8 and this is the cube. | ||
| + | :<math>\scriptstyle{\color{blue}{x^3=\frac{288}{36}=8}}</math> | ||
| + | |style="text-align:right;"|והוא שתחלק רפ"ח על ל"ו שעולה מזה ח' וככה יבא להיות המעוקב | ||
| + | |- | ||
| + | | | ||
| + | :The thing is its cube root, which is a cube root of 8; it is 2. | ||
| + | :<math>\scriptstyle{\color{blue}{x=\sqrt[3]{x^3}=\sqrt[3]{8}=2}}</math> | ||
| + | |style="text-align:right;"|והדבר יבא להיות שרשו המעוקב שהוא שרש מעוקב מח' שהוא ב‫' | ||
| + | |- | ||
| + | | | ||
| + | :You assumed the first number, or say the smaller, is 3 things and the thing is 2, so 3 things are 6; this is the first number. | ||
| + | :<math>\scriptstyle{\color{blue}{a=3x=3\sdot2=6}}</math> | ||
| + | |style="text-align:right;"|ואתה הנחת שהמספר ראשון או אמור הקטון היה ג' דברים והדבר יבא להיות ב' א"כ ג' דברים יהיו ו' וככה היה המספר ראשון | ||
| + | |- | ||
| + | | | ||
| + | :The second number is 4 things and the thing is 2, so 4 things are 8; this is the second number. | ||
| + | :<math>\scriptstyle{\color{blue}{b=4x=4\sdot2=8}}</math> | ||
| + | |style="text-align:right;"|והמספר השני היה ד' דברי' והדבר הוא ב' א"כ ד' דברים יהיו ח' וככה הוא המספר השני | ||
| + | |- | ||
| + | |If the number of the cubes has no cube root, and you wish to know how much are the first and the second numbers that are 3 things and 4 things: | ||
| + | |style="text-align:right;"|ואם המספר אשר יבא להיות המעוקב לא יהיה לו שרש מעוקב ורצית לדעת כמו יבא להיות או לשוות המספר ראשון והשני שהם ג' דברים וד' דברי‫' | ||
| + | |- | ||
| + | | | ||
| + | :You should multiply 3 by a cube root of 8 this way: | ||
| + | |style="text-align:right;"|הנך צריך להכות ג' בשרש מעוקב מח' בזה האופן | ||
| + | |- | ||
| + | | | ||
| + | :Convert 3 into a cube root; you get a cube root of 27. | ||
| + | |style="text-align:right;"|השב ג' לשרש מעוקב ויהיה לך שרש מעוקב מכ"ז | ||
| + | |- | ||
| + | | | ||
| + | :Multiply it by a cube root of 8; it is a cube root of 216. | ||
| + | |style="text-align:right;"|וזה תכה בשרש מעוקב מח' ויעשה שרש מעוקב מרי"ו | ||
| + | |- | ||
| + | | | ||
| + | :The cube root of 216, which is 6, is equal to the first number. | ||
| + | :<math>\scriptstyle{\color{blue}{a=3x=3\sdot\sqrt[3]{8}=\sqrt[3]{27}\sdot\sqrt[3]{8}=\sqrt[3]{216}=6}}</math> | ||
| + | |style="text-align:right;"|ושרש מעוקב מרי"ו שהוא ו' {{#annot:term|429,1247|V3XA}}יבא לשוות ה{{#annotend:V3XA}}מספר הראשון | ||
| + | |- | ||
| + | | | ||
| + | :The other number that is 4 things: | ||
| + | |style="text-align:right;"|ואח"כ המספר האחר שהיה ד' דברים | ||
| + | |- | ||
| + | | | ||
| + | :We convert it into a cube root; you get a cube root of 64. | ||
| + | |style="text-align:right;"|השיבנו אל שרש מעוקב ויהיה לך שרש מעוקב מס"ד | ||
| + | |- | ||
| + | | | ||
| + | :Multiply it by a cube root of 8; it is a cube root of 512, which is 8, and this is the second number. | ||
| + | :<math>\scriptstyle{\color{blue}{b=4x=4\sdot\sqrt[3]{8}=\sqrt[3]{64}\sdot\sqrt[3]{8}=\sqrt[3]{512}=8}}</math> | ||
| + | |style="text-align:right;"|וזה תכה בשרש מעוקב מח' שיבא שרש מעוקב מתקי"ב שהוא ח' וככה הוא המספר השני | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== Chapter 8 ==== | ||
| + | |||
| + | |style="width:45%;text-align:right;"|<big>הפרק הח‫'</big> | ||
| + | |- | ||
| + | |When cubes are equal to things: | ||
| + | :<math>\scriptstyle ax^3=bx</math> | ||
| + | |style="text-align:right;"|כאשר המעוקבים יהיו שוים אל הדברי‫' | ||
| + | |- | ||
| + | |The things should be divided by the cubes. | ||
| + | |style="text-align:right;"|צריך לחלק הדברי' על המעוקבי‫' | ||
| + | |- | ||
| + | |The square root of the result is equal to the thing. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{x=\sqrt{\frac{b}{a}}}}</math> | ||
| + | |style="text-align:right;"|והעולה מזה הנה שרשו המרובע שוה הדבר | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:b=2a;a²b=9b|618|ZaKZ}}Find me two numbers such that the one is double the other; | ||
| + | :and when the smaller is multiplied by itself, and the product is multiplied by the second number, it yields the same as the second, i.e. the larger, multiplied by 9. | ||
| + | :I ask: how much will each number be? | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle b=2a\\\scriptstyle a^2\sdot b=9b\end{cases}</math> | ||
| + | |style="text-align:right;"|תמצא לי שני מספרים שהאחד יהיה {{#annot:term|387,1230|HqHc}}כפל ה{{#annotend:HqHc}}שני<br> | ||
| + | וכשהוכה הקטון בעצמו ואותה ההכאה תוכה במספר השני יעשה כמו השני רצו' הגדול מוכה על ט‫'<br> | ||
| + | אשאל כמה יבא להיות כל אחד מהמספרים{{#annotend:ZaKZ}} | ||
| + | |- | ||
| + | | | ||
| + | :This is its rule: | ||
| + | |style="text-align:right;"|זהו הכלל שלו | ||
| + | |- | ||
| + | | | ||
| + | :Suppose the first number is a thing [<math>\scriptstyle{\color{blue}{a=x}}</math>], so the second should be two things [<math>\scriptstyle{\color{blue}{b=2x}}</math>], which is its double. | ||
| + | |style="text-align:right;"|תניח שהמספר הראשון יהיה דבר אחד א"כ והשני צריך להיות ב' דברים א"כ יהיה כפלו | ||
| + | |- | ||
| + | | | ||
| + | :Now, multiply the smaller number, which is one thing, by itself; the result is one square. | ||
| + | :<math>\scriptstyle{\color{blue}{x\sdot x=x^2}}</math> | ||
| + | |style="text-align:right;"|עתה תכה המספר הקטון בעצמו שהוא דבר אחד ועלה א' צינסו | ||
| + | |- | ||
| + | | | ||
| + | :I multiply this product, which is one square, by the second number, which is 2 things; the result is 2 cubes. Keep them for one side of the equation. | ||
| + | :<math>\scriptstyle{\color{blue}{x^2\sdot2x=2x^3}}</math> | ||
| + | |style="text-align:right;"|וזאת ההכאה שהוא א' צינסו אכנה במספר השני שהוא ב' דברים שעולה ב' מעוקבי' ושמור זה לחלק אחד מן ההשואה | ||
| + | |- | ||
| + | | | ||
| + | :Now, multiply the second number, which is 2 things, by 9; the result is 18 things and this is the other side of the equation. | ||
| + | :<math>\scriptstyle{\color{blue}{2x\sdot9=18x}}</math> | ||
| + | |style="text-align:right;"|ועתה תכה המספר השני שהוא ב' דברים בט' ועולה י"ח דברים וככה יהיה החלק האחר מההשואה | ||
| + | |- | ||
| + | | | ||
| + | :You have 2 cubes equal 18 things. | ||
| + | :<math>\scriptstyle{\color{blue}{2x^3=18x}}</math> | ||
| + | |style="text-align:right;"|ויהיה לך ב' מעוקבים שוים אל י"ח דברים | ||
| + | |- | ||
| + | | | ||
| + | :Pursue the above said rule: | ||
| + | |style="text-align:right;"|עתה תרדוף כפי הכלל האמור | ||
| + | |- | ||
| + | | | ||
| + | :Divide the things by the cubes, which is 18 by 2; the result is 9. | ||
| + | |style="text-align:right;"|תחלק הדברים על המעוקבים שהוא י"ח על ב' ויעלה מזה ט‫' | ||
| + | |- | ||
| + | | | ||
| + | :The root of 9 is equal to the thing. The root is 3 and this is the first number. | ||
| + | :<math>\scriptstyle{\color{blue}{a=x=\sqrt{\frac{18}{2}}=\sqrt{9}=3}}</math> | ||
| + | |style="text-align:right;"|ושרש זה הט' יבא להיות שוה הדבר וזה השרש יהיה ג' וככה הוא המספר הראשון | ||
| + | |- | ||
| + | | | ||
| + | :The second should be double the first, so it is six. | ||
| + | :<math>\scriptstyle{\color{blue}{b=2x=2\sdot3=6}}</math> | ||
| + | |style="text-align:right;"|והשני צריך להיות {{#annot:term|387|QOoq}}פי שנים מ{{#annotend:QOoq}}הראשון אם כן יבא להיות ששה | ||
| + | |- | ||
| + | |If the number has no root, meaning the quotient of the number of the things divided by the number of the cubes: | ||
| + | |style="text-align:right;"|ואם המספר לא היה לו שרש רצוני העולה בחלוק הדברי' עם המעוקבים | ||
| + | |- | ||
| + | |Multiply the result by 4 and the root of this product is the second number, because it should be its double. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{b=2x=2\sdot\sqrt{\frac{b}{a}}=\sqrt{4\sdot\frac{b}{a}}}}</math> | ||
| + | |style="text-align:right;"|תכה מה שעולה בד' ושרש אותו הסך יבא להיות המספר השני מפני שצריך להיות כפלו | ||
| + | |- | ||
| + | |If it is its thrice, it should be multiplied by 9. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{3x=3\sdot\sqrt{\frac{b}{a}}=\sqrt{9\sdot\frac{b}{a}}}}</math> | ||
| + | |style="text-align:right;"|ואם יהיה ג' דמיוניו היה צריך לכפלו או להכותו על ט‫' | ||
| + | |- | ||
| + | |And so on, the numbers should be converted to roots as you saw above. | ||
| + | |style="text-align:right;"|וכן לדמיוני אלו המספרי' בהשיב המספרים אל שרשים כאשר הראית למעלה | ||
| + | |- | ||
| + | |Know that this chapter is of the nature of the second: | ||
| + | |style="text-align:right;"|ודע כי זה הפרק הוא מטבע השני כי ככה שוה | ||
| + | |- | ||
| + | |When cubes are equal to things it is the same as when squares are equal to numbers. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{ax^3=bx\longleftrightarrow ax^2=b}}</math> | ||
| + | |style="text-align:right;"|כאשר המעוקבים יהיו שוים אל הדברים כמו שהוא כאשר הצינסי יהיו שוים אל המספרים | ||
| + | |- | ||
| + | |The reason is that when dividing all the equation by things: | ||
| + | |style="text-align:right;"|והסבה בזה היא כי לחלוק או {{#annot:term|784,2518|Kn1N}}לבקע{{#annotend:Kn1N}} ההשואה על הדברים | ||
| + | |- | ||
| + | |The cubese become squares. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{ax^3\div x=ax^2}}</math> | ||
| + | |style="text-align:right;"|המעוקבי' יצאו צינסי | ||
| + | |- | ||
| + | |The things become numbers. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{bx\div x=b}}</math> | ||
| + | |style="text-align:right;"|והדברים יצאו מספרים | ||
| + | |- | ||
| + | |Since, when multiplying a thing by a square the result is a cube. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{x\sdot x^2=x^3}}</math> | ||
| + | |style="text-align:right;"|מפני כי להכות דבר בצינסו יצא מעוקב | ||
| + | |- | ||
| + | |When multiplying a number by a thing the result is thing[s]. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{x\sdot b=bx}}</math> | ||
| + | |style="text-align:right;"|ובהכות מספר בדבר יגיע דבר | ||
| + | |- | ||
| + | |Therefore, when dividing cubes by things, the result is squares. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{ax^3\div x=ax^2}}</math> | ||
| + | |style="text-align:right;"|א"כ לחלק המעוקבים על דברי' יעלה ממנו צינסי | ||
| + | |- | ||
| + | |When dividing things by things, the result is numbers. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{bx\div x=b}}</math> | ||
| + | |style="text-align:right;"|ולחלוק דברי' על דברי' יגיעו מספרים | ||
| + | |- | ||
| + | |Thus, it is returned to the second chapter. | ||
| + | |style="text-align:right;"|ויהיה כבר שב אל הפרק השני | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== Chapter Nine ==== | ||
| + | |||
| + | |style="width:45%;text-align:right;"|<big>הפרק התשיעי</big> | ||
| + | |- | ||
| + | |When cubes are equal to squares: | ||
| + | :<math>\scriptstyle ax^3=bx^2</math> | ||
| + | |style="text-align:right;"|כאשר המעוקבי' יהיו שוים אל הצינסי | ||
| + | |- | ||
| + | |The squares should be divided by the cubes and the result is equal to the thing. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{x=\frac{b}{a}}}</math> | ||
| + | |style="text-align:right;"|צריך לחלק הצינסי על המעוקבים והעולה ממנו שוה הדבר | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:a/b=¾;(ab)·(a+b)=a²|618|bWjS}}Find me two numbers such that the one is a part of the other as 3 is of 4; | ||
| + | :and when the first is multiplied by the second, and the product is multiplied by the sum of both numbers together, it yields the same as the product of the larger by itself. | ||
| + | :I ask: how much will each number be? | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle a:b=3:4\\\scriptstyle\left(a\sdot b\right)\sdot\left(a+b\right)=a^2\end{cases}</math> | ||
| + | |style="text-align:right;"|תמצא לי שני מספרים שיהיה חלק האחד מהאחר כמו שהוא ג' מד‫'<br> | ||
| + | וכאשר הוכה הראשון בשני ואותה ההכאה תוכה על מקובץ שני המספרי' יחד יעשה כמו הכאת הגדול בעצמו<br> | ||
| + | אשאל כמה יבא להיות כל אחד מהמספרי‫'{{#annotend:bWjS}} | ||
| + | |- | ||
| + | | | ||
| + | :This is its rule: | ||
| + | |style="text-align:right;"|זהו הכלל שלו | ||
| + | |- | ||
| + | | | ||
| + | :Suppose one of the numbers is three things [<math>\scriptstyle{\color{blue}{a=3x}}</math>] and the other is four things [<math>\scriptstyle{\color{blue}{b=4x}}</math>] | ||
| + | |style="text-align:right;"|תניח שא' מהמספרי' יהיה ג' דברים והשני ד' דברי‫' | ||
| + | |- | ||
| + | | | ||
| + | :Now, multiply 3 things by 4 things; the result is 12 squares. | ||
| + | |style="text-align:right;"|עתה תכה ג' דברי' בד' דברים שעולה י"ב צינסי | ||
| + | |- | ||
| + | | | ||
| + | :Multiply this product by the sum of the two numbers, which is 7 things; the result is 84 cubes. Keep them. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(3x\sdot4x\right)\sdot\left(3x+4x\right)=12x^2\sdot7x=84x^3}}</math> | ||
| + | |style="text-align:right;"|וזאת ההכאה תכה בשני המספרים מקובצים יחד שהם ז' דברים שעולים פ"ד מעוקבי' ושמרם | ||
| + | |- | ||
| + | | | ||
| + | :Multiply the greater, which is 4 things, by itself; the result is 16 squares and this product is equal to 84 cubes. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(4x\right)^2=16x^2=84x^3}}</math> | ||
| + | |style="text-align:right;"|עתה תכה הגדול בעצמו שהוא ד' דברים שעולה י"ו צינסי וזאת ההכאה היא שוה לפ"ד מעוקבי‫' | ||
| + | |- | ||
| + | | | ||
| + | :Pursue the previously mentioned rule: | ||
| + | |style="text-align:right;"|עתה תרדוף כפי הכלל האמור קודם | ||
| + | |- | ||
| + | | | ||
| + | :Divide the squares by the cubes, which is 16 squares by 84 cubes; the result is 4 parts of 21 and this is equal to the thing. | ||
| + | :<math>\scriptstyle{\color{blue}{x=\frac{16}{84}=\frac{4}{21}}}</math> | ||
| + | |style="text-align:right;"|תחלק הצינסי על המעוקבים שהם י"ו צינסי על פ"ד מעוקבים ויעלה ד' חלקי' מכ"א וככה שוה הדבר | ||
| + | |- | ||
| + | | | ||
| + | :If we wish to know how much is the first part: you assumed the first part is 3 things and the thing is 4 parts of 21, so 3 things are 12 parts of 21, which is 4 parts of 7; this is the first number. | ||
| + | :<math>\scriptstyle{\color{blue}{a=3x=3\sdot\frac{4}{21}=\frac{12}{21}=\frac{4}{7}}}</math> | ||
| + | |style="text-align:right;"|ואם רצינו לדעת כמה יבא להיות החלק הראשון אתה הנחת שהחלק הראשון היה ג' דברים והדבר הוא ד' חלקים מכ"א<br> | ||
| + | א"כ ג' דברים יהיו י"ב חלקים מכ"א שיבא להיות ד' חלקים מז' וככה הוא המספר הראשון | ||
| + | |- | ||
| + | | | ||
| + | :You assumed the second is 4 things and the thing is 4 parts of 21, so 4 things are 16 parts of 21; this is the second number. | ||
| + | :<math>\scriptstyle{\color{blue}{b=4x=4\sdot\frac{4}{21}=\frac{16}{21}}}</math> | ||
| + | |style="text-align:right;"|והשני אתה הנחת שהיה ד' דברים והדבר שוה ד' חלקי' מכ"א<br> | ||
| + | א"כ ד' דברים יהיו י"ו חלקים מכ"א וככה הוא המספר השני | ||
| + | |- | ||
| + | |Know that this equation can be returned to the first chapter. | ||
| + | |style="text-align:right;"|ודע כי זאת ההשואה אפש' להשיבה אל הפרק הראשון | ||
| + | |- | ||
| + | |The reason is that when we divide the whole equation by the squares: | ||
| + | |style="text-align:right;"|וסבת זה היא זאת נחלק או בבקע כל ההשואה על הצינסי | ||
| + | |- | ||
| + | |The cubes become things. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{ax^3\div x^2=ax}}</math> | ||
| + | |style="text-align:right;"|המעוקבי' ישובו אל דברים | ||
| + | |- | ||
| + | |The squares become numbers. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{bx^2\div x^2=b}}</math> | ||
| + | |style="text-align:right;"|והצינסי ישובו אל מספרי‫' | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== Chapter Ten ==== | ||
| + | |||
| + | |style="width:45%;text-align:right;"|<big>הפרק העשירי</big> | ||
| + | |- | ||
| + | |When cubes are equal to squares of squares: | ||
| + | :<math>\scriptstyle bx^3=ax^4</math> | ||
| + | |style="text-align:right;"|כאשר המעוקבים יהיו שוים אל הצינסי מצינסי | ||
| + | |- | ||
| + | |The cubes should be divided by the squares of squares and the result is equal to the thing. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{x=\frac{b}{a}}}</math> | ||
| + | |style="text-align:right;"|צריך לחלק המעוקבים על הצינסי מצינסי ומה שיעלה מזה ככה שוה הדבר | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:a/b=⅘;(ab)²=a²b|618|Ukgo}}Find me two numbers such that the first is a part of the second as 4 is of 5; | ||
| + | :and when the first is multiplied by the second, and this product is multiplied by itself, it yields the same as the product of the first by itself, then that product is multiplied by the second number. | ||
| + | :I ask: how much will each number be? | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle a:b=4:5\\\scriptstyle\left(a\sdot b\right)^2=a^2\sdot b\end{cases}</math> | ||
| + | |style="text-align:right;"|תמצא לי שני {{#annot:term|994,1277|lK1I}}מספרים מתיחסים{{#annotend:lK1I}} שיהיה חלק הראשון מהשני כמו שהוא ד' מה‫'<br> | ||
| + | וכשיוכה הראשון בשני ואותה ההכאה תוכה בעצמה ויעשה כמו שעושה הראשון מוכה בעצמו ואותה ההכאה תוכה במספר השני<br> | ||
| + | אשאל כמה יבא להיות כל אחד מהמספרים{{#annotend:Ukgo}} | ||
| + | |- | ||
| + | | | ||
| + | :Follow its rule: | ||
| + | |style="text-align:right;"|תעשה כמו שרוצה כללו זה | ||
| + | |- | ||
| + | | | ||
| + | :Suppose the first is four things [<math>\scriptstyle{\color{blue}{a=4x}}</math>] and the second is five things [<math>\scriptstyle{\color{blue}{b=5x}}</math>]. | ||
| + | |style="text-align:right;"|תניח שהראשון יהיה ד' דברים והשני יהיה ה' דברי‫' | ||
| + | |- | ||
| + | | | ||
| + | :Now, multiply 4 things by 5 things; the result is 20 squares. | ||
| + | :<math>\scriptstyle{\color{blue}{4x\sdot5x=20x^2}}</math> | ||
| + | |style="text-align:right;"|עתה תכה ד' דברים בה' דברי' שעולה כ' צינסי | ||
| + | |- | ||
| + | | | ||
| + | :Multiply this product, which is 20 squares, by itself; the result is 400 squares of squares. Keep it. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(20x^2\right)^2=400x^4}}</math> | ||
| + | |style="text-align:right;"|וזאת ההכאה מכ' צינסי תכה בעצמה שעולה ת' צינסי מצינסי ושמור | ||
| + | |- | ||
| + | | | ||
| + | :Now, multiply the first part, which is 4 things, by itself; the result is 16 squares. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(4x\right)^2=16x^2}}</math> | ||
| + | |style="text-align:right;"|עתה תכה החלק הראשון בעצמו שהוא ד' דברים ועולה י"ו צינסי | ||
| + | |- | ||
| + | | | ||
| + | :Multiply 16 squares by the second number, which is 5 things; the result is 80 cubes and this product is equal to 400 squares of squares. | ||
| + | :<math>\scriptstyle{\color{blue}{16x^2\sdot5x=80x^3=400x^4}}</math> | ||
| + | |style="text-align:right;"|ואלו הי"ו צינסי תכה במספר השני שהוא ה' דברי' ויעלה פ' מעוקבי' וזאת ההכא' שוה אל ת' צינסי מצינסי | ||
| + | |- | ||
| + | | | ||
| + | :Pursue the above mentioned rule: | ||
| + | |style="text-align:right;"|ועתה תרדוף כפי הכלל האמור למעלה | ||
| + | |- | ||
| + | | | ||
| + | :Divide the cubes by the squares of squares, which is 80 by 400; the result is [a fifth] and this is the thing. | ||
| + | :<math>\scriptstyle{\color{blue}{x=\frac{80}{400}={\color{red}{\frac{1}{5}}}}}</math> | ||
| + | |style="text-align:right;"|תחלק המעוקבי' על הצינסי מצינסי שהוא פ' על ת' ויעלה ה' וככה שוה הדבר | ||
| + | |- | ||
| + | | | ||
| + | :You assumed the first number is 4 things and the thing is a fifth, so 4 things are 4 parts of 5; this is the first number. | ||
| + | :<math>\scriptstyle{\color{blue}{a=4x=4\sdot\frac{1}{5}=\frac{4}{5}}}</math> | ||
| + | |style="text-align:right;"|ואתה הנחת שהמספר הראשון היה ד' דברי' והדבר הוא א' חומש<br> | ||
| + | א"כ ד' דברים יהיו ד' חלקי' מה' וככה הוא המספר הראשון | ||
| + | |- | ||
| + | | | ||
| + | :The second number is 5 things and 5 things are 5-fifths, which is one; this is the second number. | ||
| + | :<math>\scriptstyle{\color{blue}{b=5x=5\sdot\frac{1}{5}=\frac{5}{5}=1}}</math> | ||
| + | |style="text-align:right;"|והמספר השני היה ה' דברי' וה' דברים שוים ה' חמישיות שהם אחד וככה הוא המספר השני | ||
| + | |- | ||
| + | |Know that this equation can be returned to the first chapter: | ||
| + | |style="text-align:right;"|ודע כי זאת ההשואה אפש' להשיבה אל הפרק הראשון | ||
| + | |- | ||
| + | |Because, when the cubes are divided by cubes, they become numbers. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{bx^3\div x^3=b}}</math> | ||
| + | |style="text-align:right;"|מפני כי {{#annot:term|784,2518|mVRl}}בבקעו{{#annotend:mVRl}} המעוקבי' על המעוקבים {{#annot:term|875,1566|lGob}}יבאו להיות{{#annotend:lGob}} מספרים | ||
| + | |- | ||
| + | |The squares of squares become things. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{ax^4\div x^3=ax}}</math> | ||
| + | |style="text-align:right;"|והצינסי מצינסי יבאו להיות דברים | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== Chapter 11 ==== | ||
| + | |||
| + | |style="width:45%;text-align:right;"|<big>הפרק הי"א</big> | ||
| + | |- | ||
| + | |When squares of squares are equal to numbers: | ||
| + | :<math>\scriptstyle ax^4=c</math> | ||
| + | |style="text-align:right;"|כאשר צינסי מצינסי יהיו שוים אל מספרים | ||
| + | |- | ||
| + | |The numbers should be divided by the squares of squares. | ||
| + | |style="text-align:right;"|צריך לחלק המספרים על הצינסי מצינסי | ||
| + | |- | ||
| + | |Extract a root of a root of the result and this is the thing. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{x=\sqrt[4]{\frac{c}{a}}}}</math> | ||
| + | |style="text-align:right;"|ומהעולה מזה {{#annot:term|795|rOMz}}תקח שרש שרשו{{#annotend:rOMz}} והוא יהיה הדבר | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:(a·⅔a)²=36|618|IqWv}}Find me a number, such that when it is multiplied by its two thirds, and the product is multiplied by itself, it yields 36. | ||
| + | :I ask: how much will the number be? | ||
| + | :<math>\scriptstyle\left[a\sdot\left(\frac{2}{3}\sdot a\right)\right]^2=36</math> | ||
| + | |style="text-align:right;"|תמצא לי מספר אחד שכשיוכה בשני שלישיו ועולה שני שלישי צינסו והעולה יוכה בעצמו | ||
| + | יעשה ל"ו<br> | ||
| + | אשאל כמה יבא להיות המספר{{#annotend:IqWv}} | ||
| + | |- | ||
| + | |Its rule: | ||
| + | |style="text-align:right;"|זהו הכלל שלו | ||
| + | |- | ||
| + | | | ||
| + | :defining the number as a thing <math>\scriptstyle{\color{blue}{a=x}}</math> | ||
| + | |style="text-align:right;"|תניח שהמספר יהיה דבר אחד | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{x\sdot\left(\frac{2}{3}\sdot x\right)=\frac{2}{3}x^2}}</math> | ||
| + | |style="text-align:right;"|עתה תכה דבר אחד בשני שלישיו ועולה שני שלישי צינסו | ||
| + | |- | ||
| + | | | ||
| + | ::::<math>\scriptstyle{\color{blue}{\left(\frac{2}{3}x^2\right)^2}}</math> | ||
| + | |style="text-align:right;"|ועתה תכה ב' שלישי צינסו על עצמם | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle\frac{4}{9}x^4=36</math> | ||
| + | |style="text-align:right;"|ועולה ד' תשיעיות מצינסו דצינסו שהם שוים ל"ו מספרי‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Pursuing the above rule: | ||
| + | |style="text-align:right;"|עתה תרדוף כפי הכלל האמור למעלה | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{x^2=\frac{36}{\frac{4}{9}}=81}}</math> | ||
| + | |style="text-align:right;"|תחלק המספרים על הצינסי מצינסי דהיינו ל"ו על ד' תשיעיות ו{{#annot:term|875,1566|aTvl}}יבא מזה{{#annotend:aTvl}} פ"א וככה יבא להיות הצינסי מצינסי | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{a=x=\sqrt[4]{81}=3}}</math> | ||
| + | |style="text-align:right;"|ושרש השרש מפ"א יבא להיות הדבר דהיינו המספר הנשאל אשר הנחת היותו דבר אחד וזה שרש השרש יגיע להיות ג' וככה הוא המספר | ||
| + | |- | ||
| + | |This is almost similar to the nature of the second chapter, except that here the root of the root is extracted while in the second chapter only one root is extracted. | ||
| + | |style="text-align:right;"|ודע כי זה כמעט דומה לטבע הפרק השני רק ש{{#annot:term|795|Kjmi}}לוקח שרש השרש{{#annotend:Kjmi}} והשני לוקח שרש אחד לבד | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== Chapter 12 ==== | ||
| + | |||
| + | |style="width:45%;text-align:right;"|<big>הפרק הי"ב</big> | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|עוד רודף באופן אחר דהיינו | ||
| + | |- | ||
| + | |When squares of squares are equal to things: | ||
| + | :<math>\scriptstyle ax^4=bx</math> | ||
| + | |style="text-align:right;"|כאשר הצינסי מצינסי יהיו שוים אל הדברי‫' | ||
| + | |- | ||
| + | |The things should be divided by the squares of squares. | ||
| + | |style="text-align:right;"|צריך לחלק הדברים על הצינסי מצינסי | ||
| + | |- | ||
| + | |The cube root of the result is equal to the thing. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{x=\sqrt[3]{\frac{b}{a}}}}</math> | ||
| + | |style="text-align:right;"|והעולה מזה הנה שרשו המעוקב יהיה שוה הדבר | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:a²·¾a²=6a|618|GJDy}}Find me a number, such that when it is multiplied by itself, and the product is multiplied by its three quarters, it yields the same as 6 times the number. | ||
| + | :I ask: how much will this number be? | ||
| + | :<math>\scriptstyle a^2\sdot\frac{3}{4}a^2=6a</math> | ||
| + | |style="text-align:right;"|תמצא לי מספר אחד שכאשר הוכה בעצמו ומה שיעלה יוכה ב'''שני שלישיו''' יעשה כמו ו' דמיוני המספר האמור<br> | ||
| + | אשאל כמה יהיה המספר האמור{{#annotend:GJDy}} | ||
| + | |- | ||
| + | |Following its rule: | ||
| + | |style="text-align:right;"|תעשה כמו שאומ' זה הכלל שלו | ||
| + | |- | ||
| + | | | ||
| + | :defining the number as a thing <math>\scriptstyle{\color{blue}{a=x}}</math> | ||
| + | |style="text-align:right;"|תניח שהמספר יהיה דבר אחד | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{x\sdot x=x^2}}</math> | ||
| + | |style="text-align:right;"|ותכהו בעצמו ויהיה א' צינסו | ||
| + | |- | ||
| + | | | ||
| + | ::::<math>\scriptstyle{\color{blue}{x^2\sdot\frac{3}{4}x^2=\frac{3}{4}x^4}}</math> | ||
| + | |style="text-align:right;"|וזה א' צינסו תכהו בשלשת חלקיו רצוני בג' רביעי צינסו ועולה ג' רביעי צינסו מצינסו ושמרם | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{6\sdot x=6x}}</math> | ||
| + | |style="text-align:right;"|ועתה תכה המספר שהוא ו' בדבר אחד ועושה ו' דברים | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle6x=\frac{3}{4}x^4</math> | ||
| + | |style="text-align:right;"|ואלו ו' דברים הם שוים אל ג' רביעי' צינסו מצינסו | ||
| + | |- | ||
| + | | | ||
| + | ::Pursuing the above rule: | ||
| + | |style="text-align:right;"|עתה תרדוף כפי הכלל האמור למעלה | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{x=\sqrt[3]{\frac{6}{\frac{3}{4}}}=\sqrt[3]{8}=2}}</math> | ||
| + | |style="text-align:right;"|והוא שאתה צריך לחלק הדברים על הצינסו דהיינו ו' בג' רביעי' ויגיע לך ח‫'<br> | ||
| + | ושרש המעוקב מח' יבא להיות הדבר שהוא המספר אשר הנחת היותו הדבר<br> | ||
| + | וזה השרש המעוקב יבא להיות ב' וככה הוא המספר | ||
| + | |- | ||
| + | |The [equation in this] chapter can be restored to chapter seven: | ||
| + | |style="text-align:right;"|ודע כי זה הפרק אפש' להשיבו אל הפרק השביעי | ||
| + | |- | ||
| + | | | ||
| + | *<math>\scriptstyle x^4\div x=x^3</math> | ||
| + | |style="text-align:right;"|מפני כי לבקע צינסו מצינס' על דבר {{#annot:term|875,1231|EMxT}}יצא ממנו{{#annotend:EMxT}} מעוקב | ||
| + | |- | ||
| + | | | ||
| + | *<math>\scriptstyle bx\div x=b</math> | ||
| + | |style="text-align:right;"|ודבר על דבר יצא ממנו מספר | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== Chapter 13 ==== | ||
| + | |||
| + | |style="width:45%;text-align:right;"|<big>הפרק הי"ג</big> | ||
| + | |- | ||
| + | |When squares of squares are equal to squares of squares: | ||
| + | :<math>\scriptstyle ax^4=bx^2</math> | ||
| + | |style="text-align:right;"|עוד תדע כאשר הצינסי מצינסי יהיו שוים אל הצינסי | ||
| + | |- | ||
| + | |The the whole equation, i.e. the squares should be divided by the squares of squares. | ||
| + | |style="text-align:right;"|צריך לחלק כל ההשואה דהיינו הצינסי על הצינסי מצינסי | ||
| + | |- | ||
| + | |The square root of the result is equal to the thing. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{x=\sqrt{\frac{b}{a}}}}</math> | ||
| + | |style="text-align:right;"|והעולה מזה שרשו המרובע ישוה הדבר | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:a/b=⅗;(ab)²=a²|618|0dhl}}Find me two numbers such that the first is a part of the second as 3 is of 5; | ||
| + | :and when the smaller is multiplied by the greater and the product is multiplied by itself, it yields the same as the product of the greater by itself. | ||
| + | :I ask: how much will each number be? | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle a:b=3:5\\\scriptstyle\left(a\sdot b\right)^2=a^2\end{cases}</math> | ||
| + | |style="text-align:right;"|תמצא לי שני מספרים שיהיה חלק הראשון מהשני כמו שהוא ג' מה‫'<br> | ||
| + | וכאשר הוכה הקטון בגדול ומה שיעלה יוכה בעצמו יעשה כמו הכאת הגדול בעצמו<br> | ||
| + | אשאל כמה כל אחד מהמספרים{{#annotend:0dhl}} | ||
| + | |- | ||
| + | |Its rule: | ||
| + | |style="text-align:right;"|זהו כללו | ||
| + | |- | ||
| + | | | ||
| + | :defining: | ||
| + | :*the first number as three things <math>\scriptstyle{\color{blue}{a=3x}}</math> | ||
| + | |style="text-align:right;"|תניח שהמספר הראשון יהיה ג' דברים | ||
| + | |- | ||
| + | | | ||
| + | :*the other as five things <math>\scriptstyle{\color{blue}{b=5x}}</math> | ||
| + | |style="text-align:right;"|והאחר ה' דברים | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{3x\sdot5x=15x^2}}</math> | ||
| + | |style="text-align:right;"|עתה תכה הראשון בשני שזהו ג' דברים בה' דברים עושים ט"ו צינסי | ||
| + | |- | ||
| + | | | ||
| + | ::::<math>\scriptstyle{\color{blue}{\left(15x^2\right)^2=225x^4}}</math> | ||
| + | |style="text-align:right;"|וזאת ההכאה רצוני ט"ו צינסי תכה בעצמה ועולה רכ"ה צינסי מצינסי ושמרם | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{\left(5x\right)^2}}</math> | ||
| + | |style="text-align:right;"|עתה תכה החלק הגדול בעצמו שהוא ה' דברים | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle25x^2=225x^4</math> | ||
| + | |style="text-align:right;"|ועולה כ"ה צינסי שהם שוים אל רכ"ה צינסי | ||
| + | |- | ||
| + | | | ||
| + | ::Pursuing the above rule: | ||
| + | |style="text-align:right;"|עתה תרדוף כפי הכלל האמור למעלה | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{x^2=\frac{25}{225}=\frac{1}{9}}}</math> | ||
| + | |style="text-align:right;"|תחלק הצינס' על הצינסי מצינסי דהיינו כ"ה על רכ"ה שעולה מזה א' תשיעית וכן שוה הצינסו | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{x=\sqrt{\frac{1}{9}}=\frac{1}{3}}}</math> | ||
| + | |style="text-align:right;"|ושרש א' תשיעית יבא להיות הדבר והשרש הזה יבא להיות א' שלישי' וכן הוא הדבר | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{a=3x=3\sdot\frac{1}{3}=1}}</math> | ||
| + | |style="text-align:right;"|ואתה הנחת המספר הראשון היותו ג' דברי' והדבר הוא א' שלישי<br> | ||
| + | א"כ ג' דברי' יהיו אחד שלם וכן הוא המספר הראשון | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{b=5x=5\sdot\frac{5}{3}=1+\frac{2}{3}}}</math> | ||
| + | |style="text-align:right;"|והמספר השני הונח היותו ה' דברים והדבר הוא א' שלישי<br> | ||
| + | א"כ יהיו ה' שלישים שהם א' וב' שלישי' וכן הוא המספר השני | ||
| + | |- | ||
| + | |If <math>\scriptstyle\frac{b}{a}</math> has no root: | ||
| + | |style="text-align:right;"|ואם {{#annot:term|783,2086|zngW}}המספרי' המגיעי' לך בחלוק{{#annotend:zngW}} הצינסי על הצינסי מצינסי {לא היה להם שרש | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{a=3x=\sqrt{3^2\sdot\frac{1}{9}}=\sqrt{9\sdot\frac{1}{9}}=\sqrt{1}=1}}</math> | ||
| + | |style="text-align:right;"|ובקשת לדעת כמה הוא ג' דברים תכה ג' בעצמו עושה ט‫'<br> | ||
| + | ולכן תכה מה שעלה שהוא א' תשיעי' בט' ועושה א' שלם<br> | ||
| + | ושרש א' שהוא א' יבא להיות המספר הראשון | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{b=5x=\sqrt{5^2\sdot\frac{1}{9}}=\sqrt{25\sdot\frac{1}{9}}=\sqrt{\frac{25}{9}}=\frac{5}{3}=1+\frac{2}{3}}}</math> | ||
| + | |style="text-align:right;"|והמספר השני אשר הונח ה' דברי' תכה ה' בשרש א' תשיעית שעולה שרש כ"ה תשיעיות<br> | ||
| + | שהוא ה' שלישיו‫'<br> | ||
| + | דהיינו א' וב' שלישי וכן הוא המספר השני | ||
| + | |- | ||
| + | |This equation can be restored to chapter two, by dividing all the equation by a square: | ||
| + | |style="text-align:right;"|ודע כי זאת ההשואה אפש' להשיבה אל הפרק השני מפני כי בחלק ההשואה על הצינסי | ||
| + | |- | ||
| + | | | ||
| + | *<math>\scriptstyle x^4\div x^2=x^2</math> | ||
| + | |style="text-align:right;"|מצינסי יצא צינסי | ||
| + | |- | ||
| + | | | ||
| + | *<math>\scriptstyle bx^2\div x^2=b</math> | ||
| + | |style="text-align:right;"|והצינסי מצינסי יצאו מספרים | ||
| + | |- | ||
| + | |<math>\scriptstyle{\color{blue}{225x^4=25x^2\quad/\div x^2\longrightarrow\quad225x^2=25}}</math> which is restored to chapter two. | ||
| + | |style="text-align:right;"|א"כ רכ"ה צינסי יבאו שוים אל כ"ה צינסי סקיסאנדי שהוא כ"ה מספרים ויבא מושב אל הפרק השני רכ"ה צינסי שוים אל כ"ה מספרים | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== Chapter 14 ==== | ||
| + | |||
| + | |style="width:45%;text-align:right;"|<big>פרק י"ד</big> | ||
| + | |- | ||
| + | |When cubes and squares are equal to things: | ||
| + | :<math>\scriptstyle ax^3+bx^2=cx</math> | ||
| + | |style="text-align:right;"|כאשר המעוקבים והצינסי יהיו שוים אל הדברי‫' | ||
| + | |- | ||
| + | |The whole equation should be divided by the number of the cubes. | ||
| + | |style="text-align:right;"|צריך לחלק כל ההשואה על כמות המעוקבים | ||
| + | |- | ||
| + | |Then, [the number of] the squares should be halved, i.e. in two equal parts. | ||
| + | |style="text-align:right;"|ואח"כ לחלק הצינסי לחצי רצו' לשני חלקים שוים | ||
| + | |- | ||
| + | |Multiply one of the halves by itself. | ||
| + | |style="text-align:right;"|ואחד מאותם החצאים תכה בעצמו | ||
| + | |- | ||
| + | |Add the product to [the number of] the things. | ||
| + | |style="text-align:right;"|ואותה ההכאה תחבר אל הדברים | ||
| + | |- | ||
| + | |The root of this sum minus the other half of [the number of] the squares is equal to the thing and the thing is a root of the square. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{x=\sqrt{\left(\frac{1}{2}\sdot\frac{b}{a}\right)^2+\frac{c}{a}}-\left(\frac{1}{2}\sdot\frac{b}{a}\right)}}</math> | ||
| + | |style="text-align:right;"|ושרש זה הסך פחות המחצית האחר מהצינסי יבא לשוות הדבר<br> | ||
| + | והדבר יבא להיות שרש ממה שהוא הצינסו | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:(a²·a)+b²=12½c|618|iH47}}Find me three numbers, such that the first is a part of the second as 2 is of 3, and the second is of the third as 3 is of 4. | ||
| + | :If the first is multiplied by itself, then the product is multiplied by the first number, and the second number is multiplied by itself, when both products are summed together, its yields the same as the product of the third number by 12 and a half. | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle a:b=2:3\\\scriptstyle b:c=3:4\\\scriptstyle\left(a^2\sdot a\right)+b^2=c\sdot\left(12+\frac{1}{2}\right)\end{cases}</math> | ||
| + | |style="text-align:right;"|והנה המשל תמצא לי שלשה מספרים שיהיה חלק הראשון מהשני כמו שהוא ב' מג‫'<br> | ||
| + | והשני יהיה מהשלישי כמו שהוא ג' בד‫'<br> | ||
| + | ותכה הראשון בעצמו ואח"כ יוכה העולה במספר הראשון בעצמו ואח"כ יוכה המספר השני בעצמו וכשיחוברו יחד שתי אלו ההכאות | ||
| + | יעשה כמו הכאת המספר השלישי בי"ב וחצי<br> | ||
| + | אשאל כמה יבא להיות כל אחד מהמספרים{{#annotend:iH47}} | ||
| + | |- | ||
| + | |Its rule: | ||
| + | |style="text-align:right;"|זהו הכלל שלו | ||
| + | |- | ||
| + | | | ||
| + | :defining: | ||
| + | :*the first number as two things <math>\scriptstyle{\color{blue}{a=2x}}</math> | ||
| + | |style="text-align:right;"|תניח שהמספר הראשון היה ב' דברים | ||
| + | |- | ||
| + | | | ||
| + | :*the second as three things <math>\scriptstyle{\color{blue}{b=3x}}</math> | ||
| + | |style="text-align:right;"|והשני ג' דברי‫' | ||
| + | |- | ||
| + | | | ||
| + | :*the third as four things <math>\scriptstyle{\color{blue}{c=4x}}</math> | ||
| + | |style="text-align:right;"|והשלישי ד' דברי‫' | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{\left(2x\right)^2=4x^2}}</math> | ||
| + | |style="text-align:right;"|עתה תכה המספר הראשון בעצמו רצוני ב' דברים ועולה ד' צינסי | ||
| + | |- | ||
| + | | | ||
| + | ::::<math>\scriptstyle{\color{blue}{4x^2\sdot2x=8x^3}}</math> | ||
| + | |style="text-align:right;"|עתה תכה זאת ההכאה שהיא ד' צינסי במספר הראשון שהיא ב' דברים ועולה ח' מעוקבים ושמרם | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{\left(3x\right)^2=9x^2}}</math> | ||
| + | |style="text-align:right;"|אח"כ תכה המספר השני בעצמו שעולה ט' צינסי | ||
| + | |- | ||
| + | | | ||
| + | ::::<math>\scriptstyle{\color{blue}{8x^3+9x^2}}</math> | ||
| + | |style="text-align:right;"|ו{{#annot:term|178,1165|tvJI}}חברם על{{#annotend:tvJI}} הח' מעוקבים אשר שמרת ויהיה לך ח' מעוקבי' וט' צינסי ושמור זה הסך בעד אחד מהחלקים | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{4x\sdot\left(12+\frac{1}{2}\right)=50x}}</math> | ||
| + | |style="text-align:right;"|אח"כ תכה המספר השלישי דהיינו ד' דברי' בי"ב וחצי ועולה נ' דברי' שהם החלק האחר מההשואה | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle8x^3+9x^2=50x</math> | ||
| + | |style="text-align:right;"|א"כ ח' מעוקבי' וט' צינסי הם שוים אל נ' דברים | ||
| + | |- | ||
| + | | | ||
| + | ::Pursuing the above rule: | ||
| + | |style="text-align:right;"|עתה תרדוף כפי הכלל האמור למעלה | ||
| + | |- | ||
| + | | | ||
| + | :<span style=color:Green>'''Normalization''':</span> <math>\scriptstyle{\color{blue}{8x^3+9x^2=50x\quad/\div8}}</math> | ||
| + | |style="text-align:right;"|והוא שאתה צריך לחלק כל דבר בכמו מה שהם המעוקבים רצוני כל ההשואה על ח‫' | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle x^3+\left(1+\frac{1}{8}\right)x^2=\left(6+\frac{1}{4}\right)x</math> | ||
| + | |style="text-align:right;"|ויעלה לך א' מעוקב וא' צינסו וא' שמינית שוים אל ו' דברים ורביע | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|עתה צריך אתה לחלק הצינסי לחצי ויעלה ט' חלקים מי"ו | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ואלו הט' חלקים מי"ו תכם בעצמם ויעלה פ"א חלקים מרנ"ו | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|וזאת ההכאה תחבר על הדברי' שהם ו' ורביע ויהיה לך ו' וקמ"ה חלקים מרנ"ו | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ושרש אלו הו' וקמ"ה חלקים מרנ"ו פחות המחצית האחר מהצינסי שאומ' פחות ט' חלקים מי"ו יבא לשוות הדבר | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ושרש זה הוא ב' וט' חלקים מי"ו | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ומזה צריך להוציא המחצית האחד מהצינסי שהוא ט' חלקים מי"ו וישאר ב' וכן שוה הדבר | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle x&\scriptstyle=\sqrt{\left[\frac{1}{2}\sdot\left(1+\frac{1}{8}\right)\right]^2+\left(6+\frac{1}{4}\right)}-\left[\frac{1}{2}\sdot\left(1+\frac{1}{8}\right)\right]=\sqrt{\left(\frac{9}{16}\right)^2+\left(6+\frac{1}{4}\right)}-\frac{9}{16}=\sqrt{\frac{81}{256}+\left(6+\frac{1}{4}\right)}-\frac{9}{16}\\&\scriptstyle=\sqrt{6+\frac{145}{256}}-\frac{9}{16}=\left(2+\frac{9}{16}\right)-\frac{9}{16}=2\\\end{align}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{a=2x=4}}</math> | ||
| + | |style="text-align:right;"|ואתה הנחת היות המספר הראשון ב' דברי' א"כ יבא להיות המספר הראשון ד‫' | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{b=3x=6}}</math> | ||
| + | |style="text-align:right;"|והמספר השני שמת היותו ג' דברי' שיבא להיות ו‫' | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{c=4x=8}}</math> | ||
| + | |style="text-align:right;"|והשלישי הנחת היותו ד' דברים א"כ היה ח‫' | ||
| + | |- | ||
| + | |These are the numbers required above. | ||
| + | |style="text-align:right;"|וכן יבאו להיות המספרי' המבוקשים למעלה | ||
| + | |- | ||
| + | |The [equation in] this chapter can be restored to chapter 4, by dividing all the equation by a thing <math>\scriptstyle{\color{blue}{8x^3+9x^2=50x\quad/\div x\longrightarrow\quad8x^2+9x=50}}</math> | ||
| + | |style="text-align:right;"|ודע כי זה הפרק אפש' להביאו אל הפרק הרביעי מפני כי {{#annot:term|784,2519|1It4}}להבקיע{{#annotend:1It4}} כל ההשואה על דבר יגיע ח' צינסי וט' דברים שוים אל נ' דברים | ||
| + | |- | ||
| + | |which can be divided then by the number of the squares <math>\scriptstyle{\color{blue}{8x^2+9x=50\quad/\div8\longrightarrow\quad x^2+\left(1+\frac{1}{8}\right)x=6+\frac{1}{4}}}</math> | ||
| + | |style="text-align:right;"|או נאמר א' צינסו וא' שמינית וא' דבר שוים לו' דברים ורביע בהיות נחלק כפי מה שהם הצינסי | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== Chapter 15 ==== | ||
| + | |||
| + | |style="width:45%;text-align:right;"|<big>פרק ט"ו</big> | ||
| + | |- | ||
| + | |When cubes and things are equal to squares: | ||
| + | :<math>\scriptstyle ax^3+cx=bx^2</math> | ||
| + | |style="text-align:right;"|כאשר המעוקבים והדברים יהיו שוים אל הצינסי | ||
| + | |- | ||
| + | |The whole equation should be divided by the numbers of cubes. | ||
| + | |style="text-align:right;"|צריך לחלק כל ההשואה על כמו שהם המעוקבים | ||
| + | |- | ||
| + | |Then, [the number of] the squares should be halved. | ||
| + | |style="text-align:right;"|ואח"כ לחלק הצינסי לחצאים | ||
| + | |- | ||
| + | |Multiply one of the halves by itself. | ||
| + | |style="text-align:right;"|ואחד מהחצאי' תכהו בעצמו | ||
| + | |- | ||
| + | |Subtract the number of the things from this product. | ||
| + | |style="text-align:right;"|ומאותה ההכאה תוציא כמות הדברים | ||
| + | |- | ||
| + | |We extract the root of the remainder. | ||
| + | |style="text-align:right;"|ומן הנשאר נקח שרשו | ||
| + | |- | ||
| + | |We add it to the other half of [the number of] the squares and this is equal to the thing. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{x=\left(\frac{1}{2}\sdot\frac{b}{a}\right)+\sqrt{\left(\frac{1}{2}\sdot\frac{b}{a}\right)^2-\frac{c}{a}}}}</math> | ||
| + | |style="text-align:right;"|ונוסיפהו על המחצית האחר מהצינסי וככה ישוה הדבר | ||
| + | |- | ||
| + | | | ||
| + | *<math>\scriptstyle x=\left(\frac{1}{2}\sdot\frac{b}{a}\right)-\sqrt{\left(\frac{1}{2}\sdot\frac{b}{a}\right)^2-\frac{c}{a}}</math> | ||
| + | |style="text-align:right;"|ופעמים מה תצטרך לומ' שהדבר שוה מחצית הצינסי פחות השרש מאשר הנשאר | ||
| + | |- | ||
| + | | | ||
| + | *<math>\scriptstyle x=\left(\frac{1}{2}\sdot\frac{b}{a}\right)+\sqrt{\left(\frac{1}{2}\sdot\frac{b}{a}\right)^2-\frac{c}{a}}</math> | ||
| + | |style="text-align:right;"|ופעמים מה יהיה אפשר לענות שהדבר ישוה מחצית הצינסי ויותר שרש הנשאר | ||
| + | |- | ||
| + | |According to the way of the fifth chapter. | ||
| + | |style="text-align:right;"|או פחות שרש הנשאר כפי האופן מהפרק החמישי | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:a+b=10, (a-b)²·a=20¼a|619|qbxm}}Divide ten into two parts, such that when the difference between the one and the other is multiplied by itself, and this product is multiplied by the greater part, it yields the same as the product of the greater part by twenty and a quarter. | ||
| + | :I ask: how much will each one of the parts be? | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle a+b=10\\\scriptstyle\left(a-b\right)^2\sdot a=a\sdot\left(20+\frac{1}{4}\right)\end{cases}</math> | ||
| + | |style="text-align:right;"|והנני עושה לך מזה המשל עשה לי מחלקים מעשרה שני חלקים באופן שכאשר יוכה ההבדל שבין האחד אל האחר בעצמו וזאת ההכאה תוכה בחלק הגדול יעשה כמו הכאת החלק הגדול בעשרים | ||
| + | ורביע<br> | ||
| + | אשאל כמה יבא להיות כל אחד מהחלקים{{#annotend:qbxm}} | ||
| + | |- | ||
| + | |Its rule: | ||
| + | |style="text-align:right;"|זהו כללו | ||
| + | |- | ||
| + | | | ||
| + | :defining: | ||
| + | :*one of the parts as a thing <math>\scriptstyle{\color{blue}{a=x}}</math> | ||
| + | |style="text-align:right;"|תניח שאחד מהחלקי' יהיה דבר אחד | ||
| + | |- | ||
| + | | | ||
| + | :*the other will be ten minus a thing <math>\scriptstyle{\color{blue}{b=10-x}}</math> | ||
| + | |style="text-align:right;"|והאחר יבא להיות עשרה פחות דבר אחד | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{x-\left(10-x\right)=2x-10}}</math> | ||
| + | |style="text-align:right;"|עתה תקח {{#annot:term|997,1651|Vd6Q}}ההבדל שהוא בין זה לזה{{#annotend:Vd6Q}} שיבא להיות ב' דברים פחות עשרה | ||
| + | |- | ||
| + | |When we want to restore it to the first aforementioned answer of this chapter. | ||
| + | |style="text-align:right;"|ברצותנו להשיבה אל זה הפרק בעד התשובה הראשונה האמורה למעלה | ||
| + | |- | ||
| + | | | ||
| + | ::::<math>\scriptstyle{\color{blue}{\left(2x-10\right)^2=4x^2+{\color{red}{100}}-40x}}</math> | ||
| + | |style="text-align:right;"|עתה תכה זה ההבדל בעצמו דהיינו ב' דברים פחות עשרה ועולה ד' צינסי ועשרה דברים פחות מ' דברים | ||
| + | |- | ||
| + | | | ||
| + | :::::<math>\scriptstyle{\color{blue}{\left(4x^2+100-40x\right)\sdot x=4x^3+100x-40x^2}}</math> | ||
| + | |style="text-align:right;"|וזאת ההכאה תכה עוד בחלק הגדול אשר אני עושה שהוא דבר אחד כדי להשיב החשבון אל זה הפרק ויעלה ד' מעוקבי' וק' דברים פחות מ' צינסו ושמור זה בעבור אחד מהחלקי‫' | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{x\sdot\left(20+\frac{1}{4}\right)=\left(20+\frac{1}{4}\right)x}}</math> | ||
| + | |style="text-align:right;"|עתה תכה החלק הגדול אשר עשינוהו היותו דבר אחד בכ' ורביע ויעלה כ' דברי' ורביע וזה יהיה החלק השני מההשואה | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle4x^3+100x-40x^2=\left(20+\frac{1}{4}\right)x</math> | ||
| + | |style="text-align:right;"|ויהיה לך ד' מעוקבי' וק' דברי' פחות מ' צינסי שוים לכ' דברים ורביע | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{4x^3+100x-40x^2=\left(20+\frac{1}{4}\right)x\quad/+40x^2}}</math> | ||
| + | |style="text-align:right;"|עתה תחבר מ' צינסי שהם גורעים לאחד מהחלקים ובדומה לזה תחבר עוד אל החלק האחר | ||
| + | |- | ||
| + | |As said above that instead subtracting one needs to add, so one needs to add to one side the same as the other. | ||
| + | |style="text-align:right;"|כאשר נאמ' לך לפנים שבמקום הגורע צריך להוסיף וכן צריך להוסיף על החלק האחד כמו אל האחר | ||
| + | |- | ||
| + | |Since you added to the one as to the other, you need to subtract the smaller quantity of the things from each side. | ||
| + | |style="text-align:right;"|ואחר שהוספת על האחד כמו לאחר צריך אתה להוציא הכמות הקטון מהדברי' מכל אחד מהחלקי‫' | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle4x^3+\left(79+\frac{3}{4}\right)x=40x^2</math> | ||
| + | |style="text-align:right;"|וישאר לך ד' מעוקבי' וע"ט דברי' וג' רביעים שוים אל מ' צינסי | ||
| + | |- | ||
| + | | | ||
| + | :<span style=color:Green>'''Normalization''':</span> <math>\scriptstyle{\color{blue}{4x^3+\left(79+\frac{3}{4}\right)x=40x^2\quad/\div4}}</math> | ||
| + | |style="text-align:right;"|עתה תחלק כל ההשואה הזאת השניה כאמור למעלה דהיינו לחלוק בכמות המעוקבים שיבא לחלוק על ד‫' | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle x^3+\left(19+\frac{15}{16}\right)x=10x^2</math> | ||
| + | |style="text-align:right;"|ויבא א' מעוקב וי"ט דברים וט"ו חלקים מי"ו שוים אל עשרה צינסי | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|עתה תחלק הצינסי לחצי ויעלה ה‫' | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ואלו הה' תכם בעצמם ועולה כ"ה | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ומאלו הכ"ה תוציא כמות שהם י"ט וט"ו חלקים מי"ו וישאר ה' וחלק א' מי"ו | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ושרש ה' וא' חלק מי"ו שהוא ב' ורביע תוסיף על המחצית האחד מהצינסי שהוא על הה' ויהיה לך שהדבר יבא להיות ז' ורביע | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle a=x&\scriptstyle=\left(\frac{1}{2}\sdot10\right)+\sqrt{\left(\frac{1}{2}\sdot10\right)^2-\left(19+\frac{15}{16}\right)}=5+\sqrt{5^2-\left(19+\frac{15}{16}\right)}=5+\sqrt{25-\left(19+\frac{15}{16}\right)}\\&\scriptstyle=5+\sqrt{5+\frac{1}{16}}=5+\left(2+\frac{1}{4}\right)=7+\frac{1}{4}\\\end{align}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{a=5+\sqrt{5+\frac{1}{16}}}}</math> | ||
| + | |style="text-align:right;"|או אם תרצה אמור ה' ויותר שרש מן ה' וחלק א' מי"ו כפי מה שנתן הפרק האמור וכן יבא להיות החלק הגדול | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{b=10-x=10-\left(7+\frac{1}{4}\right)=2+\frac{3}{4}}}</math> | ||
| + | |style="text-align:right;"|והאחר יבא להיות הנשאר עד עשרה שהוא ב' וג' רביעים | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{b=5-\sqrt{5+\frac{1}{16}}}}</math> | ||
| + | |style="text-align:right;"|או אם תרצה אמור ה' פחות שרש ה' וחלק מי"ו והוא החלק הקטן | ||
| + | |- | ||
| + | |This equation is restored to chapter 5, by dividing all the equation by a thing, which yields the answer by the first way so that the thing is a number plus a root | ||
| + | :<math>\scriptstyle ax^3+cx=bx^2\quad/\div x\longrightarrow\quad ax^2+c=bx\longrightarrow\quad x=A+\sqrt{B}</math> | ||
| + | |style="text-align:right;"|ודע כי זאת ההשואה שהיא מעוקבים ודברי' שוים לצינסי ישוב אל הפרק החמישי בחלוק על דבר כל ההשואה ותעשה לך התשובה באופן הראשון היות הדבר מספר ויותר שרש | ||
| + | |- | ||
| + | |Another calculation whose answer is by the second way of this chapter, which is a number minus a root. | ||
| + | |style="text-align:right;"|עוד רצוני לתת לך חשבון אחר שתשובתו תהיה מזה הפרק באופן השני שהוא מספר פחות שרש | ||
| + | |- | ||
| + | |I will give you another question for chapter 15: | ||
| + | |style="text-align:right;"|עוד אניח לך שאלה אחרת בעד פרק ט"ו האמור | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:a+b=10, (a-b)²·b=20¼b|619|BCIc}}Divide ten into two parts, such that when the difference between the two parts is multiplied by itself, and this product is multiplied by the smaller part, it yields the same as the product of the smaller part by twenty and a quarter. | ||
| + | :I ask: how much will each one of the parts be? | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle a+b=10\\\scriptstyle\left(a-b\right)^2\sdot b=b\sdot\left(20+\frac{1}{4}\right)\end{cases}</math> | ||
| + | |style="text-align:right;"|עשה לי מעשרה שני חלקים באופן שכאשר יוכה ההבדל שבין שני החלקים בעצמו ואותה ההכאה תכה בחלק הקטון יעשה כמו הכאת החלק הקטון בכ' ורביע<br> | ||
| + | אשאל כמה יבא להיות כל אחד מהחלקים{{#annotend:BCIc}} | ||
| + | |- | ||
| + | |Following its rule: | ||
| + | |style="text-align:right;"|תעשה כמו שאומ' כללו | ||
| + | |- | ||
| + | | | ||
| + | :defining: | ||
| + | :*one of the parts as a thing <math>\scriptstyle{\color{blue}{b=x}}</math> | ||
| + | |style="text-align:right;"|תניח שאחד מהחלקי' יהיה דבר אחד | ||
| + | |- | ||
| + | | | ||
| + | :*the other will be ten minus a thing <math>\scriptstyle{\color{blue}{a=10-x}}</math> | ||
| + | |style="text-align:right;"|והאחר יבא להיות עשרה פחות דבר אחד | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{\left(10-x\right)-x=10-2x}}</math> | ||
| + | |style="text-align:right;"|עתה תקח ההבדל שבין האחד אל האחר שהוא עשרה פחות ב' דברים | ||
| + | |- | ||
| + | |If we want to restore it now to the second answer of this chapter. | ||
| + | |style="text-align:right;"|ואם רצינו להשיבה אל זה הפרק בתשובה השנית עתה | ||
| + | |- | ||
| + | | | ||
| + | ::::<math>\scriptstyle{\color{blue}{\left(10-2x\right)^2=100+4x^2-40x}}</math> | ||
| + | |style="text-align:right;"|תכה זה ההבדל בעצמו שהוא עשרה פחות ב' דברים ועולה ק' מספרים וד' צינסי פחות מ' דברים | ||
| + | |- | ||
| + | | | ||
| + | :::::<math>\scriptstyle{\color{blue}{\left(100+4x^2-40x\right)\sdot x=100x+4x^3-40x^2}}</math> | ||
| + | |style="text-align:right;"|וזאת ההכאה מוכת על החלק הקטון אשר אנחנו עשינו היותו דבר אחד כדי להשיבו אל התשובה השנית ועולה השנית ועולה ק' דברים וד' מעוקבים פחות מ' צינסי ו{{#annot:term|459,1237|xPel}}שמרם בעבור{{#annotend:xPel}} שאחד מהחלקים מההשואה | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{x\sdot\left(20+\frac{1}{4}\right)=\left(20+\frac{1}{4}\right)x}}</math> | ||
| + | |style="text-align:right;"|עתה תכה החלק הקטון אשר עשינו היותו דבר אחד בכ' ורביע ויעלה כ' דברים ורביע וכן יהיה החלק השני מההשואה | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle100x+4x^3-40x^2=\left(20+\frac{1}{4}\right)x</math> | ||
| + | |style="text-align:right;"|ויהיה לך מאה דברי' וד' מעוקבי' פחות מ' צינסי שוים אל כ' דברים ורביע | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{100x+4x^3-40x^2=\left(20+\frac{1}{4}\right)x\quad/+40x^2}}</math> | ||
| + | |style="text-align:right;"|עתה תוסיף מ' צינסי אשר פוחתים מאחד החלקים ואם תעשה כפי מה שהראית לפנים תוסיפם לחלק האחר גם כן ותוציא הכמות הקטון מהדברים מכל אחד מהחלקים שהוא כ' דברים ורביע | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle\left(79+\frac{3}{4}\right)x+4x^3=40x^2</math> | ||
| + | |style="text-align:right;"|וישאר ע"ט דברים וג' רביעים וד' מעוקבים שוים אל מ' צינסי | ||
| + | |- | ||
| + | | | ||
| + | :<span style=color:Green>'''Normalization''':</span> <math>\scriptstyle{\color{blue}{\left(79+\frac{3}{4}\right)x+4x^3=40x^2\quad/\div4}}</math> | ||
| + | |style="text-align:right;"|עתה תחלק כל זאת ההשואה כפי מה שנאמ' לך לפנים דהיינו בכמו שהם המעוקבים שיבאו {{#annot:term|784,1226|rNoY}}להחלק על{{#annotend:rNoY}} ד‫' | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle x^3+\left(19+\frac{15}{16}\right)x=10x^2</math> | ||
| + | |style="text-align:right;"|ויבא א' מעוקב וי"ט דברים וט"ו חלקים מי"ו שוי' אל עשרה צינסו | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|עתה תחלק הצינסי לחצאים ויבא מזה ה‫' | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ותכה ה' על עצמו ועושה מזה כ"ה | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ומאלו הכ"ה תוציא הדברי' שהם י"ט וט"ו חלקי' מי"ו וישאר ה' וחלק א' מי"ו | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ושרשם שהוא ב' ורביע תוציא מהחצי האחר מהצינסי שהוא ה‫'<br> | ||
| + | ויהיה לך היות הדבר ב' וג' רביעי‫' | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle b=x&\scriptstyle=\left(\frac{1}{2}\sdot10\right)-\sqrt{\left(\frac{1}{2}\sdot10\right)^2-\left(19+\frac{15}{16}\right)}=5-\sqrt{5^2-\left(19+\frac{15}{16}\right)}=5-\sqrt{25-\left(19+\frac{15}{16}\right)}\\&\scriptstyle=5-\sqrt{5+\frac{1}{16}}=5-\left(2+\frac{1}{4}\right)=2+\frac{3}{4}\\\end{align}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{b=5-\sqrt{5+\frac{1}{16}}}}</math> | ||
| + | |style="text-align:right;"|או אם תרצה תאמר ה' פחות שרש ה' וחלק מי"ו כפי מה שהפרק השיב לו וככה יבא להיות החלק הקטון | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{a=10-x=10-\left(2+\frac{3}{4}\right)=7+\frac{1}{4}}}</math> | ||
| + | |style="text-align:right;"|והאחר יבא להיות הנשאר עד עשרה שהוא ז' ורביע | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{a=5+\sqrt{5+\frac{1}{16}}}}</math> | ||
| + | |style="text-align:right;"|או תאמ' ה' ושרש ה' וחלק מי"ו והוא החלק הגדול | ||
| + | |- | ||
| + | |The answer is done so that the root is subtracted in the thing as the stated method of the chapter. | ||
| + | |style="text-align:right;"|ונעשתה התשובה להיות הדבר פחות שרש כפי אופן הפרק אשר אמרתי לך | ||
| + | |- | ||
| + | |I also want to show you how the chapter can assign the thing a number and a root and a number minus a root by that same calculation of chapter 15: | ||
| + | |style="text-align:right;"|עוד רצוני להראות לך איך הפרק האמור אפש' ליתן הדבר מספר ושרש ומספר פחות שרש בחשבון אחד ממש עוד בעבור הפרק הט"ו האמור | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:a+b=10, (ab)·a=21a|619|Wwvi}}Divide ten into two parts, such that when the first part is multiplied by the second, and this product is multiplied by the first, it yields the same as the product of the first by 21. | ||
| + | :I ask: how much will each one of the parts be? | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle a+b=10\\\scriptstyle\left(a\sdot b\right)\sdot a=21a\end{cases}</math> | ||
| + | |style="text-align:right;"|עשה לי מעשרה שני חלקים באופן שכאשר יוכה החלק הראשון בשני וההכאה ההיא תוכה בראשון יעשה כמו הכאת הראשון בכ"א<br> | ||
| + | אשאל כמה יבא להיות כל אחד מהחלקים האמורים{{#annotend:Wwvi}} | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{a=x}}</math> | ||
| + | |style="text-align:right;"|זהו כללו תניח שהחלק הראשון יהיה דבר אחד | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{b=10-x}}</math> | ||
| + | |style="text-align:right;"|והשני יבא להיות עשרה פחות דבר אחד | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{{\color{red}{x\sdot\left(10-x\right)}}=10x-x^2}}</math> | ||
| + | |style="text-align:right;"|ועולה עשרה דברים פחות א' צינסו | ||
| + | |- | ||
| + | | | ||
| + | ::::<math>\scriptstyle{\color{blue}{\left(10x-x^2\right)\sdot x=10x^2-x^3}}</math> | ||
| + | |style="text-align:right;"|וזאת ההכאה תכה עוד בחלק הראשון שהוא דבר אחד ועולה עשרה צינסי פחות א' מעוקב ושמור | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{x\sdot21}}</math> | ||
| + | |style="text-align:right;"|אח"כ תכה החלק הראשון שהוא דבר אחד בכ"א | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle21x=10x^2-x^3</math> | ||
| + | |style="text-align:right;"|ועולה כ"א דברים והם שוים אל עשרה צינסי פחות א' מעוקב | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{21x=10x^2-x^3\quad/+x^3}}</math> | ||
| + | |style="text-align:right;"|עתה תתן המעוקב שהוא פחות לכל אחד מהחלקים | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle x^3+21x=10x^2</math> | ||
| + | |style="text-align:right;"|ויהיה לך א' מעוקב וכ"א דברים שוים אל עשרה צינסי | ||
| + | |- | ||
| + | | | ||
| + | :<span style=color:Green>'''Normalization''':</span> <math>\scriptstyle{\color{blue}{x^3+21x=10x^2\quad/\div1}}</math> | ||
| + | |style="text-align:right;"|עתה צריך אתה לחלק כל ההשואה בכמו שהם המעוקבים שהוא אחד | ||
| + | |- | ||
| + | | | ||
| + | ::the result is the same: [<math>\scriptstyle x^3+21x=10x^2</math>] | ||
| + | |style="text-align:right;"|ועולה מזה אותו בעצמו | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|עתה עליך לחלוק הצינסי לחצאים כמו שרוצה הכלל האמור ויעלה ה‫' | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ותכה ה' בעצמו ועולה כ"ה | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ומזה הכ"ה תוציא כמות הדברי' שהם כ"א וישאר ד‫' | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ושרש זה הד' תוסיף על מחצית כמות הצינסי דהינו על ה‫'<br> | ||
| + | או להוציא זה השרש חוצה מזה הה‫' | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{a=x=\left(\frac{1}{2}\sdot10\right)\pm\sqrt{\left(\frac{1}{2}\sdot10\right)^2-21}=5\pm\sqrt{5^2-21}=5\pm\sqrt{25-21}=5\pm\sqrt{4}}}</math> | ||
| + | |- | ||
| + | |Since this calculation can be answered by addition and by subtraction, one can answer that the thing is 5 plus a root of 4, or say [that it is] 5 minus a root of 4, according to the third way of the chapter. | ||
| + | |style="text-align:right;"|מפני כי זה החשבון תוכל לענות ביותר ובפחות א"כ תוכל לענות שהדבר הוא ה' ושרש ד' או תאמר ה' פחות שרש ד' כפי האופן השלישי מהפרק האמור | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== Chapter 16 ==== | ||
| + | |||
| + | |style="width:45%;text-align:right;"|<big>פרק י"ו</big> | ||
| + | |- | ||
| + | |When squares and things are equal to cubes: | ||
| + | :<math>\scriptstyle bx^2+cx=ax^3</math> | ||
| + | |style="text-align:right;"|כאשר הצינסי והדברים יהיו שוים למעוקבים | ||
| + | |- | ||
| + | |The whole equation should be divided by the number of the cubes. | ||
| + | |style="text-align:right;"|צריך לחלק כל ההשואה על כל כמות המעוקבים | ||
| + | |- | ||
| + | |Then, the number of the squares should be halved. | ||
| + | |style="text-align:right;"|ואח"כ לחלק כמות הצינסי לחצאים | ||
| + | |- | ||
| + | |Multiply one of the halves by itself. | ||
| + | |style="text-align:right;"|ולהכות אחד מהחצאים על עצמו | ||
| + | |- | ||
| + | |Add this product to the number of the things. | ||
| + | |style="text-align:right;"|וזאת ההכאה תוסיף על כמות הדברים | ||
| + | |- | ||
| + | |Add the root of this sum to the other half of the number of the squares and it is equal to the thing, i.e. half of the number of the squares plus the root of this sum. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{x=\sqrt{\left(\frac{1}{2}\sdot\frac{b}{a}\right)^2+\frac{c}{a}}+\left(\frac{1}{2}\sdot\frac{b}{a}\right)}}</math> | ||
| + | |style="text-align:right;"|ושרש זה הסך תוסיף אל המחצית האחר מכמות הצינסי וככה יבא לשוות הדבר<br> | ||
| + | דהיינו מחצית כמות הצינסי ויותר שרש אותו הסך | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:a²·a=12a+10a²|618|FhTO}}Find me a number, such that when it is multiplied by itself, and the product is multiplied by that number, it yields the same as the product of that number by 12, and that number multiplied by itself, then this product is multiplied by ten and this is added to the product by 12. | ||
| + | :I ask: how much will the number be? | ||
| + | :<math>\scriptstyle a^2\sdot a=12a+10a^2</math> | ||
| + | |style="text-align:right;"|עשה לי החשבון תמצא לי מספר אחד שכאשר הוכה בעצמו ואותה ההכאה תוכה במספר ההוא יעשה כמו הכאת המספר האמור בי"ב ואח"כ להכות המספר האמור בעצמו ואותה ההכאה תוכה בעשרה ולחבר עם ההכאה שנעשתה בי"ב<br> | ||
| + | אשאל כמה יבא להיות המספר האמור{{#annotend:FhTO}} | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{a=x}}</math> | ||
| + | |style="text-align:right;"|זהו הכלל שלו תניח שהמספר יהיה דבר אחד | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{\left(x\sdot x\right)\sdot x=x^2\sdot x=x^3}}</math> | ||
| + | |style="text-align:right;"|ותכהו בעצמו ויהיה א' צינסו וזאת ההכאה תכה על המספר שהוא דבר אחד ועולה א' מעוקב ושמרהו בעבור חלק אחד מההשואות | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{x\sdot12=12x}}</math> | ||
| + | |style="text-align:right;"|אח"כ תכה המספר האמור בי"ב דהיינו דבר אחד בי"ב ועולה י"ב דברים | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{\left(x\sdot x\right)\sdot10=x^2\sdot10=10x^2}}</math> | ||
| + | |style="text-align:right;"|ואח"כ תשוב אל המספר האמור שהוא דבר אחד תכהו בעצמו ועלה א' צינסו<br> | ||
| + | וזה הצינסו תכהו בעשרה ועולה י' צינסו | ||
| + | |- | ||
| + | | | ||
| + | ::::<math>\scriptstyle{\color{blue}{10x^2+12x}}</math> | ||
| + | |style="text-align:right;"|ואותם תחבר עם ההכאה העשויה בי"ב שהם י"ב דברים | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle10x^2+12x=x^3</math> | ||
| + | |style="text-align:right;"|ויהיה לך עשרה צינסי וי"ב דברים שוים לא' מעוקב | ||
| + | |- | ||
| + | | | ||
| + | ::Pursuing the above rule: | ||
| + | |style="text-align:right;"|עתה תרדוף כפי הכלל האמור למעלה | ||
| + | |- | ||
| + | | | ||
| + | :<span style=color:Green>'''Normalization''':</span> <math>\scriptstyle{\color{blue}{10x^2+12x=x^3\quad/\div1}}</math> | ||
| + | |style="text-align:right;"|תחלק כל ההשואות כל כמות המעוקבים שהוא אחד | ||
| + | |- | ||
| + | | | ||
| + | ::the result is the same: [<math>\scriptstyle10x^2+12x=x^3</math>] | ||
| + | |style="text-align:right;"|ויעלה אותו בעצמו | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|עתה תחלק כמות הצינסי לחצאי' ויעלה ה‫' | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|וזה הה' תכהו בעצמו ועולה כ"ה | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|תוסיף אלו הכ"ה על כמות הדברים שהם י"ב ויהיה לך ל"ז | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ושרש אלו הל"ז תחבר אל המחצית האחר מהצינסי שהוא ה' ויהיה לך שהדבר יבא לשוות ה' ויותר שרש מל"ז וככה יבא להיות המספר האמור | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{a=x=\left(\frac{1}{2}\sdot10\right)+\sqrt{\left(\frac{1}{2}\sdot10\right)^2+12}=5+\sqrt{5^2+12}=5+\sqrt{25+12}=5+\sqrt{37}}}</math> | ||
| + | |- | ||
| + | |This equation can be restored to chapter 6, by dividing all the equation by a thing <math>\scriptstyle{\color{blue}{8x^3=6x^2+12x\quad/\div x\longrightarrow\quad8x^2=6x+12}}</math> | ||
| + | |style="text-align:right;"|ודע כי זאת ההשואה אפש' להשיבה אל הפרק הששי בחלוק על דבר כל ההשואה ויעלה ח' צינסו שוה לו' דברים וי"ב מספרי‫' | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== Chapter 17 ==== | ||
| + | |||
| + | |style="width:45%;text-align:right;"|<big>פרק י"ז</big> | ||
| + | |- | ||
| + | |When things are equal to a root of numbers: | ||
| + | :<math>\scriptstyle bx=\sqrt{c}</math> | ||
| + | |style="text-align:right;"|כאשר הדברים יהיו שוים אל שרשי המספרים | ||
| + | |- | ||
| + | |The number [of the things] should be nultiplied by itself. | ||
| + | |style="text-align:right;"|צריך להכות הכמות בעצמו | ||
| + | |- | ||
| + | |Then, the numbers should be divided by this product. | ||
| + | |style="text-align:right;"|ולחלק המספרים על ההכאה ההיא | ||
| + | |- | ||
| + | |The root of the result is equal to the thing. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{x=\sqrt{\frac{c}{b^2}}}}</math> | ||
| + | |style="text-align:right;"|ושרש העולה מזה שוה הדבר | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:a/b=⅔;4a+4b=√100|618|FMUp}}Find me two numbers such that one is a part of the other as 2 is of 3; | ||
| + | :and when each of them is multiplied by 4, and both products are summed together, they yield a root of 100. | ||
| + | :I ask: how much will each number be? | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle a:b=2:3\\\scriptstyle4a+4b=\sqrt{100}\end{cases}</math> | ||
| + | |style="text-align:right;"|תמצא לי שני מספרים שיהיה חלק האחד מהאחר כמו שהוא ב' מג‫'<br> | ||
| + | וכשיוכה כל אחד בארבעה ויקובצו אלו ההכאות יחד יעשו שרש ק‫'<br> | ||
| + | אשאל כמה יבא להיות כל מספר מהם{{#annotend:FMUp}} | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{a=2x}}</math> | ||
| + | |style="text-align:right;"|זהו הכלל שלו תניח שאחד מהמספרים יהיה ב' דברים | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{b=3x}}</math> | ||
| + | |style="text-align:right;"|והאחר יבא להיות שלשה דברים | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{2x\sdot4=8x}}</math> | ||
| + | |style="text-align:right;"|עתה תכה ב' דברים בד' ועולה ח' דברים ושמרם | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{3x\sdot4=12x}}</math> | ||
| + | |style="text-align:right;"|אח"כ תכה המספר האחד שהוא ג' דברים בד' ועולה י"ב דברים | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{8x+12x}}</math> | ||
| + | |style="text-align:right;"|עתה תחבר ח' דברים עם י"ב דברים | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle20x=\sqrt{100}</math> | ||
| + | |style="text-align:right;"|ועולים כ' דברים שהם שוים אל שרש ק‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Pursuing the above rule: | ||
| + | |style="text-align:right;"|עתה תרדוף כפי הכלל האמור למעלה | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{x=\sqrt{\frac{100}{20^2}}=\sqrt{\frac{100}{400}}=\sqrt{\frac{1}{4}}}}</math> | ||
| + | |style="text-align:right;"|תכה כמות הדברים בעצמם שהם כ' דברים ויעלה ת‫'<br> | ||
| + | עתה תחלק כמות המספרים {{#annot:term|1073,2561|yY63}}אשר נקבו שרש{{#annotend:yY63}} והם ק' ותחלק אלו הק' בהכאת כמות הדברים שהוא ת' שיבא א' רביע<br> | ||
| + | ושרש א' רביע שוה הדבר | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{a=2x=2\sdot\sqrt{\frac{1}{4}}=1}}</math> | ||
| + | |style="text-align:right;"|והמספר הראשון שמתו ב' דברים אשר יבא להיות שרש א' רביע דהיינו א' שלם וכן הוא המספר הראשון | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{b=3x=3\sdot\sqrt{\frac{1}{4}}=1+\frac{1}{2}}}</math> | ||
| + | |style="text-align:right;"|והשני הנחת היותו ג' דברים אשר יבא להיות ג' פעמים שרש א' ורביע שהוא א' וחצי וככה הוא המספר השני | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{\begin{cases}\scriptstyle a=\sqrt{1}\\\scriptstyle b=\sqrt{2+\frac{1}{4}}\end{cases}}}</math> | ||
| + | |style="text-align:right;"|א"כ הראשון יבא להיות שרש האחד והשני יבא להיות שרש ב' ורביע | ||
| + | |- | ||
| + | |If one wishes to restore this equation to one of the six chapters, it can be restored to chapter two. | ||
| + | |style="text-align:right;"|ואם רצית להשיב זאת ההשואה אל אחד מהו' פרקים דע שאפש' להשיבה אל הפרק השני | ||
| + | |- | ||
| + | |Since <math>\scriptstyle\left(\sqrt{c}\right)^2=c</math> | ||
| + | |style="text-align:right;"|מפני כי שרש מספר מוכה בשרש מספר מוכה בעצמו אי פרודושא יעשה מספר | ||
| + | |- | ||
| + | |and <math>\scriptstyle\left(x\right)^2=\left(\sqrt{x^2}\right)^2=x^2</math> | ||
| + | |style="text-align:right;"|והדבר שהוא שרש מצינסו מוכה בעצמו עושה צינסו | ||
| + | |- | ||
| + | |Therefore, <math>\scriptstyle{\color{blue}{20x=\sqrt{100}\longleftrightarrow400x^2=100}}</math> | ||
| + | |style="text-align:right;"|וא"כ ת' צינסו הם שוים אל ק' מספרים | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== Chapter 18 ==== | ||
| + | |||
| + | |style="width:45%;text-align:right;"|<big>פרק י"ח</big> | ||
| + | |- | ||
| + | |When numbers are equal to a root of things: | ||
| + | :<math>\scriptstyle c=\sqrt{bx}</math> | ||
| + | |style="text-align:right;"|עוד כאשר המספרים יהיו שוים אל שרשי הדברים | ||
| + | |- | ||
| + | |The number should be nultiplied by itself. | ||
| + | |style="text-align:right;"|צריך להכות המספר בעצמו | ||
| + | |- | ||
| + | |Then, this product should be divided by the number of the things that are the radicand; the result is equal to the thing. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{x=\frac{c^2}{b}}}</math> | ||
| + | |style="text-align:right;"|ואותה ההכאה {{#annot:term|784,1966|F78a}}תחולק ב{{#annotend:F78a}}כמות הדברים אשר נקבו בשמות והעולה מזה ישוה הדבר | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:√(9a)=12|618|TeOu}}Find me a number, such that when it is multiplied by 9, the root of the product is 12. | ||
| + | :I ask: how much will the number be? | ||
| + | :<math>\scriptstyle\sqrt{9a}=12</math> | ||
| + | |style="text-align:right;"|תמצא לי מספר אחד שכאשר יוכה בט' יהיה שרש העולה י"ב<br> | ||
| + | אשאל כמה יבא להיות המספר{{#annotend:TeOu}} | ||
| + | |- | ||
| + | |Following its rule: | ||
| + | |style="text-align:right;"|תעשה כמו שאומ' כללו זה | ||
| + | |- | ||
| + | | | ||
| + | :defining the number as a thing <math>\scriptstyle{\color{blue}{a=x}}</math> | ||
| + | |style="text-align:right;"|תניח שהמספר יהיה דבר אחד | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{x\sdot9=9x}}</math> | ||
| + | |style="text-align:right;"|ותכה דבר אחד בט' ועושה ט' דברים | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle\sqrt{9x}=12</math> | ||
| + | |style="text-align:right;"|ושרש ט' דברי' הוא שוה אל י"ב | ||
| + | |- | ||
| + | | | ||
| + | ::Pursuing the above rule: | ||
| + | |style="text-align:right;"|עתה תרדוף כפי הכלל האמור למעלה | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{x=\frac{12^2}{9}=\frac{144}{9}=16}}</math> | ||
| + | |style="text-align:right;"|תכה המספרים בעצמם שהוא י"ב על י"ב ועושה קמ"ד<br> | ||
| + | ותחלק על כמות הדברים {{#annot:term|1073,2561|NJIn}}אשר נקבו היות להם שרשים{{#annotend:NJIn}} דהיינו על ט' ויעלה מזה י"ו<br> | ||
| + | ואלו הי"ו יבא לשוות הדבר וככה הוא המספר האמור | ||
| + | |- | ||
| + | |The equation <math>\scriptstyle c=\sqrt{bx}</math> can be restored to chapter one in this way: | ||
| + | |style="text-align:right;"|ודע כי ההשואה הזאת דהיינו מספרים שוים אל שרשים דברים ישוב אל הפרק הראשון בזה האופן | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{\left(\sqrt{9x}\right)^2=9x}}</math> | ||
| + | |style="text-align:right;"|דהיינו תכה שרש ט' דברים בעצמו יעלה ט' דברי‫' | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{\left(12\right)^2=144}}</math> | ||
| + | |style="text-align:right;"|וי"ב מספרי' שהם שרש מהכאה המוכה בעצמה עושה קמ"ד מספרי‫' | ||
| + | |- | ||
| + | |Since <math>\scriptstyle\sqrt{x}=\sqrt{c}\longleftrightarrow x=c</math> | ||
| + | |style="text-align:right;"|מפני כי ככה שוה כאשר שרש הדבר שוה לשרש מספר כמו כשהדבר שוה למספר | ||
| + | |- | ||
| + | |Because, when the roots of the things equal numbers, these [numbers] are also equal to the root of other numbers [<math>\scriptstyle\sqrt{bx}=c=\sqrt{c^2}</math>]. | ||
| + | |style="text-align:right;"|מפני כי בהיות שרשי הדברי' שוים אל מספרי' מה אותם הם ג"כ שרשים למספרי' אחרי‫' | ||
| + | |- | ||
| + | |Therefore, one should only convert those numbers to roots, and by this the things will be equal to numbers. | ||
| + | |style="text-align:right;"|א"כ אין צורך רק להשיב אותם המספרי' אל שרשים ובאותו ההיות יהיה א"כ הדברי' עם המספרי‫' | ||
| + | |- | ||
| + | |Thus, it is restored to chapter one. | ||
| + | |style="text-align:right;"|ויהיה מושב אל הפרק הראשון | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== Chapter 19 ==== | ||
| + | |||
| + | |style="width:45%;text-align:right;"|<big>פרק י"ט</big> | ||
| + | |- | ||
| + | |When squares are equal to a root of numbers: | ||
| + | :<math>\scriptstyle ax^2=\sqrt{c}</math> | ||
| + | |style="text-align:right;"|כאשר הצינסי יהיו שוים אל שרשי דראמי או מספרי‫' | ||
| + | |- | ||
| + | |The number of the squares should be nultiplied by itself. | ||
| + | |style="text-align:right;"|צריך להכות כמות הצינסי בעצמם | ||
| + | |- | ||
| + | |Then, the numbers should be divided by the square, or by the product of [the number of] the squares. | ||
| + | |style="text-align:right;"|ואח"כ לחלק הדראמי או המספרי' על המרובע או בהכאת הצינסי | ||
| + | |- | ||
| + | |The root of the root of the result is equal to the thing. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{x=\sqrt[4]{\frac{c}{a^2}}}}</math> | ||
| + | |style="text-align:right;"|ושרש השרש מהעולה יבא לשוות הדבר | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:a/b=⅔;ab=√12|618|zNTn}}Find me two numbers such that one is a part of the other as 2 is of 3; | ||
| + | :and if one is multiplied by the other, it yields a root of 12. | ||
| + | :I ask: how much will each number be? | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle a:b=2:3\\\scriptstyle a\sdot b=\sqrt{12}\end{cases}</math> | ||
| + | |style="text-align:right;"|תמצא לי שני מספרים שיהיה חלק האחד מהאחר כמו שהוא ב' מג‫'<br> | ||
| + | ואם יוכה האחד באחר יעשה שרש מי"ב<br> | ||
| + | אשאל כמה יבא להיות כל אחד מהמספרים{{#annotend:zNTn}} | ||
| + | |- | ||
| + | |Following its rule: | ||
| + | |style="text-align:right;"|תעשה כמו שאומ' זה הכלל שלו | ||
| + | |- | ||
| + | | | ||
| + | :defining: | ||
| + | :*one as two things <math>\scriptstyle{\color{blue}{a=2x}}</math> | ||
| + | |style="text-align:right;"|תניח שהאחד יהיה ב' דברים | ||
| + | |- | ||
| + | | | ||
| + | :*the other will be three [things] <math>\scriptstyle{\color{blue}{b=3x}}</math> | ||
| + | |style="text-align:right;"|והאחר יהיה ג‫' | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{2x\sdot3x=6x^2}}</math> | ||
| + | |style="text-align:right;"|עתה תכה האחד באחר שהוא ב' דברים בג' דברים ויעלה ו' צינסי | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle6x^2=\sqrt{12}</math> | ||
| + | |style="text-align:right;"|ואלו הו' צינסי הם שוים אל שרש י"ב | ||
| + | |- | ||
| + | | | ||
| + | ::Pursuing the above rule: | ||
| + | |style="text-align:right;"|עתה תרדוף כפי הכלל האמור למעלה | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|תכה כמות הצינסי בעצמם והם ו' ועולה ל"ו<br> | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ועתה תחלק המספרי' {{#annot:term|1073,2561|DLNs}}אשר נקבו להיות להם שרש{{#annotend:DLNs}} והם י"ב על מרובע זה מהצינסי רצו' על ל"ו ויבא א' שליש | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ושרש השרש מזה שעלה שהיה א' שליש יבא להיות הדבר | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{x=\sqrt[4]{\frac{12}{6^2}}=\sqrt[4]{\frac{12}{36}}=\sqrt[4]{\frac{1}{3}}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :You assumed the first number is 2 things, so multiply 2 by a root of a root of a third; the result is a root of a root of 5 and a third and this is the first number. | ||
| + | :<math>\scriptstyle{\color{blue}{a=2x=2\sdot\sqrt[4]{\frac{1}{3}}=\sqrt[4]{5+\frac{1}{3}}}}</math> | ||
| + | |style="text-align:right;"|ואתה הנחת שהמספר הראשון היה ב' דברים<br> | ||
| + | א"כ תכה ב' על שרש משרש א' שליש ועולה שרש משרש ה' ושליש וככה יבא להיות המספר הראשון | ||
| + | |- | ||
| + | | | ||
| + | :You assumed the second is 3 things and the thing is a root of a root of a third; so multiply 3 by a root of a root of a third; the result is a root of a root of 27 and this is the second number. | ||
| + | :<math>\scriptstyle{\color{blue}{b=3x=3\sdot\sqrt[4]{\frac{1}{3}}=\sqrt[4]{27}}}</math> | ||
| + | |style="text-align:right;"|ואתה הנחת שהשני היה ג' דברי' והדבר הוא שרש השרש מא' ושליש<br> | ||
| + | א"כ תכה ג' בשרש השרש מא' ושליש<br> | ||
| + | ועולה שרש משרש כ"ז וככה יבא להיות המספר השני | ||
| + | |- | ||
| + | |The equation <math>\scriptstyle ax^2=\sqrt{c}</math> can be restored to chapter 11 in this way: | ||
| + | |style="text-align:right;"|ודע כי זאת ההשואה רצוני שצינסי יהיו שוים אל שרשי המספרי' אפש' להשיבה אל הפרק הי"א בזה האופן | ||
| + | |- | ||
| + | | | ||
| + | ::converting <math>\scriptstyle{\color{blue}{6x^2}}</math> into roots | ||
| + | |style="text-align:right;"|תשיב ו' צינסי אל שרשים | ||
| + | |- | ||
| + | |<math>\scriptstyle ax^2=\sqrt{c}\longleftrightarrow\sqrt{Ax^4}=\sqrt{c}\longleftrightarrow Ax^4=c</math> | ||
| + | |style="text-align:right;"|ויהיה לך שרשים מצינסי מצינסי שוים לשרשי' ממספרים ושוה כמו שהיו צינסי מצינסי שוים אל מספרי‫' | ||
| + | |- | ||
| + | |This way it is restored to chapter 11. | ||
| + | |style="text-align:right;"|ותהיה מושבת בזה האופן אל הפרק הי"א | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== Chapter 20 ==== | ||
| + | |||
| + | |style="text-align:right;"|<big>פרק כ‫'</big> | ||
| + | |- | ||
| + | |When the numbers are equal to a root of squares: | ||
| + | :<math>\scriptstyle c=\sqrt{ax^2}</math> | ||
| + | |style="width:45%;text-align:right;"|עוד כאשר המספרי' יהיו שוים אל שרשי צינסי | ||
| + | |- | ||
| + | |The numbers should be multiplied by themselves. | ||
| + | |style="text-align:right;"|צריך להכות המספרי' בעצמם | ||
| + | |- | ||
| + | |Then, this product should be divided by the number of the squares that are the radicand. | ||
| + | |style="text-align:right;"|ולחלק ההכאה ההיא בכמות הצינסי {{#annot:term|1073,2300|89gu}}הנקובים שהם להם שרש{{#annotend:89gu}} | ||
| + | |- | ||
| + | |The root of the result is equal to the thing. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{x=\sqrt{\frac{c^2}{a}}}}</math> | ||
| + | |style="text-align:right;"|ושרש העולה שוה הדבר | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:a/b=⅔;(3a+4b)√5=40|618|PB7l}}Find me two numbers such that one is a part of the other as 2 is of 3; | ||
| + | :and when the first is multiplied by 3 and the second by 4, and both products are summed together and multiplied by the root of 5, it yields 40. | ||
| + | :I ask: how much will each number be? | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle a:b=2:3\\\scriptstyle\left(3a+4b\right)\sdot\sqrt{5}=40\end{cases}</math> | ||
| + | |style="text-align:right;"|והנה המשל תמצא לי שני מספרים שיהיה חלק אחד מהם לאחר כמו שהוא ב' אל ג‫'<br> | ||
| + | וכשיוכה הראשון בשלשה והשני בארבעה ואלו שתי ההכאות מחוברים יחד ויוכה בשרש ה' יעשה מ‫'<br> | ||
| + | אשאל כמה יבא להיות כל אחד מהמספרי‫'{{#annotend:PB7l}} | ||
| + | |- | ||
| + | | | ||
| + | :This is its rule: | ||
| + | |style="text-align:right;"|זהו כללו | ||
| + | |- | ||
| + | | | ||
| + | :Suppose the first number is two things [<math>\scriptstyle{\color{blue}{a=2x}}</math>] and the other is three things [<math>\scriptstyle{\color{blue}{b=3x}}</math>]. | ||
| + | |style="text-align:right;"|תניח שהמספר הראשון יהיה ב' דברים והאחר יבא להיות ג' דברי‫' | ||
| + | |- | ||
| + | | | ||
| + | :Now, multiply the first, which is 2 things, by 3; the result is 6 things. Keep them. | ||
| + | :<math>\scriptstyle{\color{blue}{3\sdot2x=6x}}</math> | ||
| + | |style="text-align:right;"|עתה תכה הראשון שהוא ב' דברים על ג' ועולה ו' דברי' ושמרם | ||
| + | |- | ||
| + | | | ||
| + | :Then, multiply the second number, which is 3 things, by 4; the result is 12 things. | ||
| + | :<math>\scriptstyle{\color{blue}{4\sdot3x=12x}}</math> | ||
| + | |style="text-align:right;"|אח"כ תכה המספר השני שהוא ג' דברי' בד' ועולה י"ב דברי‫' | ||
| + | |- | ||
| + | | | ||
| + | :Add them to the 6 things you kept; you have 18 things. | ||
| + | :<math>\scriptstyle{\color{blue}{6x+12x=18x}}</math> | ||
| + | |style="text-align:right;"|ותחברם עם ו' דברי' אשר שמרת ויהיו לך י"ח דברי‫' | ||
| + | |- | ||
| + | | | ||
| + | :Multiply 18 things by a root of 5: | ||
| + | |style="text-align:right;"|עתה תכה י"ח דברים בשרש ה‫' | ||
| + | |- | ||
| + | | | ||
| + | :Remember that you must convert the things into roots; the result is a root of 324 squares. | ||
| + | |style="text-align:right;"|זכור כי הנך צריך להשיב י"ח דברי' אל שרשים אשר {{#annot:term|875,1566|E07H}}יבא אל{{#annotend:E07H}} שרש שכ"ד צינסי | ||
| + | |- | ||
| + | | | ||
| + | :Multiply it by a root of 5; you get a root of 1625 that is equal to 40 numbers. | ||
| + | :<math>\scriptstyle{\color{blue}{18x\sdot\sqrt{5}=\sqrt{324x^2\sdot5}=\sqrt{1620x^2}=40}}</math> | ||
| + | |style="text-align:right;"|אשר תכם בשרש ה' ויהיה לך שרש מאלף תר"כ צינסי שהם שוים אל מ' מספרים | ||
| + | |- | ||
| + | | | ||
| + | :Pursue the above mentioned rule: | ||
| + | |style="text-align:right;"|עתה תרדוף כפי הכלל האמור למעלה | ||
| + | |- | ||
| + | | | ||
| + | :Multiply the numbers that are 40 by themselves; the result is 1600. | ||
| + | |style="text-align:right;"|תכה המספרי' שהם מ' בעצמם ועולי' אלף ות"ר | ||
| + | |- | ||
| + | | | ||
| + | :Divide 1600 by the number of the squares that are the radicand, which is 1620; the result is 80 parts of 81. | ||
| + | |style="text-align:right;"|וחלק אלף ת"ר בכמות הצינסי אשר נקבו היות להם שרש שהם אלף תר"כ ויבא מזה פ' חלקים מפ"א | ||
| + | |- | ||
| + | | | ||
| + | :The root of 80 parts of 81 is equal to the thing. | ||
| + | :<math>\scriptstyle{\color{blue}{x=\sqrt{\frac{40^2}{1620}}=\sqrt{\frac{1600}{1620}}=\sqrt{\frac{80}{81}}}}</math> | ||
| + | |style="text-align:right;"|ושרש פ' חלקים מפ"א יבא לשוות הדבר | ||
| + | |- | ||
| + | | | ||
| + | :You assumed the first number is 2 things, so multiply 2 by a root of 80 parts of 81; the result is a root of 3 and 77 parts of 81 and this is the first number. | ||
| + | :<math>\scriptstyle{\color{blue}{a=2x=2\sdot\sqrt{\frac{80}{81}}=\sqrt{3+\frac{77}{81}}}}</math> | ||
| + | |style="text-align:right;"|ואתה הנחת שהמספר הראשון היה ב' דברים<br> | ||
| + | א"כ תכפול ב' בשרש פ' חלקי' מפ"א ועולה שרש ג' וע"ז חלקים מפ"א וככה יבא להיות המספר הראשון | ||
| + | |- | ||
| + | | | ||
| + | :You assumed the second number is 3 things, so multiply 3 by [a root of] 80 parts of 81; the result is a root of 8 and 72 parts of 81 and this is the second number. | ||
| + | :<math>\scriptstyle{\color{blue}{b=3x=3\sdot\sqrt{\frac{80}{81}}=\sqrt{8+\frac{72}{81}}}}</math> | ||
| + | |style="text-align:right;"|והמספר השני הנחת היותו ג' דברי‫'<br> | ||
| + | א"כ תכה ג' בפ' חלקי' מפ"א ועולה שרש ח' וע"ב חלקים מפ"א וככה יבא להיות המספר השני | ||
| + | |- | ||
| + | |Know that this equation can be returned to the second chapter: | ||
| + | |style="text-align:right;"|ודע כי זאת ההשואה אפש' להשיבה אל הפרק השני | ||
| + | |- | ||
| + | |Since a root of a square equals a root of a number is the same as a square equals a number. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\sqrt{x^2}=\sqrt{c}\longleftrightarrow x^2=c}}</math> | ||
| + | |style="text-align:right;"|מפני כי כך שוה שרש מצינסו שוה אל שרש מספר כמו צינסו שוה אל מספר | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{x=\sqrt{\frac{1600}{1620}}\longleftrightarrow x^2=\frac{1600}{1620}}}</math> | ||
| + | |style="text-align:right;"|וזה ראית באותו שחלקת שרש אלף ת"ר מספרי' בשרש אלף תר"כ צינסי | ||
| + | |- | ||
| + | |Know also that this equation can be returned to the first chapter: | ||
| + | |style="text-align:right;"|ודע עוד כי זאת ההשואה אפש' להשיבה אל הפרק הראשון | ||
| + | |- | ||
| + | |Since a root of a square is a thing, so we get a thing equals a number. | ||
| + | |style="text-align:right;"|מפני כי שרש הצינסו יבא להיות הדבר א"כ היו לנו דבר שוה אל מספר | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{\sqrt{1620x^2}=40\longleftrightarrow\sqrt{1620}x=40}}</math> | ||
| + | |style="text-align:right;"|דהיינו כל כך דברים כמו שהוא שרש אלף תר"כ מספרי' שוים אל מ' מספרי‫' | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{x=\frac{40}{\sqrt{1620}}=\sqrt{\frac{80}{81}}}}</math> | ||
| + | |style="text-align:right;"|ש{{#annot:term|783,1566|D7Be}}יבא לחלק{{#annotend:D7Be}} מ' בשרש אלף תר"כ שיבא מזה באופן החלוק בשרשים שרש פ' חלקים מפ"א וככה יבא לשוות הדבר | ||
| + | |- | ||
| + | |Solved according to the said chapter. | ||
| + | |style="text-align:right;"|ונעשה בעד הפרק האמור | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== Chapter 21 ==== | ||
| + | |||
| + | |style="text-align:right;"|<big>פרק כ"א</big> | ||
| + | |- | ||
| + | |When the cubes are equal to a root of a number: | ||
| + | :<math>\scriptstyle ax^3=\sqrt{c}</math> | ||
| + | |style="width:45%;text-align:right;"|כאשר המעוקבים יהיו שוים אל שרש מספר | ||
| + | |- | ||
| + | |The number of the cubes should be multiply by itself. | ||
| + | |style="text-align:right;"|צריך להכות כמות המעוקבים בעצמם | ||
| + | |- | ||
| + | |Then, the numbers that are the radicand should be divided by that product. | ||
| + | |style="text-align:right;"|ולחלק המספרים {{#annot:term|1073,2300|JxaB}}הנקובים שרש{{#annotend:JxaB}} באותה ההכאה | ||
| + | |- | ||
| + | |Extract the square root of the cube root of the result, or vice versa the cube root of the square root, and this is the thing. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{x=\sqrt[6]{\frac{c}{a^2}}}}</math> | ||
| + | |style="text-align:right;"|ומהעולה {{#annot:term|795|Shkx}}תקח השרש מרובע משרשו המעוקב{{#annotend:Shkx}} או בהפך {{#annot:term|2634|UL3S}}שרשו המעוקב משרשו המרובע{{#annotend:UL3S}}<br> | ||
| + | וככה יבא לשוות הדבר | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:a/b=¾;a²b=√(20¼)|618|4NoH}}Find me two numbers such that one is a part of the other as 3 is of 4; | ||
| + | :and when the one is multiplied by itself, and this product is multiplied by the second, it yields a root of 20 and a quarter. | ||
| + | :I ask: how much will each number be? | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle a:b=3:4\\\scriptstyle a^2\sdot b=\sqrt{20+\frac{1}{4}}\end{cases}</math> | ||
| + | |style="text-align:right;"|עשה לי זה החשבון תמצא לי שני מספרים שיהיה חלק האחד מהאחר כמו מה שהוא ג' מד‫'<br> | ||
| + | וכשיוכה הראשון בעצמו ואותה ההכאה תכה בשני יעשה שרש כ' ורביע<br> | ||
| + | אשאל כמה יבא להיות כל אחד מהמספרים{{#annotend:4NoH}} | ||
| + | |- | ||
| + | | | ||
| + | :Do as its rule says: | ||
| + | |style="text-align:right;"|תעשה כמו שאומ' הכלל שלו | ||
| + | |- | ||
| + | | | ||
| + | :Suppose the first number is three things [<math>\scriptstyle{\color{blue}{a=2x}}</math>] and the second is four things [<math>\scriptstyle{\color{blue}{b=4x}}</math>]. | ||
| + | |style="text-align:right;"|תניח שהמספר הראשון היה ג' דברי' והשני ד' דברי‫' | ||
| + | |- | ||
| + | | | ||
| + | :Now, multiply the first, which is 3 things, by itself; the result is 9 squares. | ||
| + | |style="text-align:right;"|עתה תכה הראשון בעצמו שהוא ג' דברים ועולה ט' צינסי | ||
| + | |- | ||
| + | | | ||
| + | :Multiply this product, which is 9 square, by the second number, which is 4 things; the result is 36 cubes and they are equal to a root of 20 and a quarter. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(3x\right)^2\sdot4x=9x^2\sdot4x=36x^3=\sqrt{20+\frac{1}{4}}}}</math> | ||
| + | |style="text-align:right;"|עתה תכה זאת ההכאה שהיא ט' צינסי במספר השני שהוא ד' דברים ועולה ל"ו מעוקבי' והם יבאו להיות שוים אל שרש כ' ורביע | ||
| + | |- | ||
| + | | | ||
| + | :Pursue the given rule: | ||
| + | |style="text-align:right;"|עתה תרדוף כפי הכלל הנתון | ||
| + | |- | ||
| + | | | ||
| + | :That is, multiply the number of the cubes by itself; the result is 1296. | ||
| + | |style="text-align:right;"|דהיינו תכה כמות המעוקבים בעצמם ועולה אלף ורצ"ו | ||
| + | |- | ||
| + | | | ||
| + | :Then, divide 20 and a quarter, which is the number that is the radicand, by 1296; the result is one part of 64. | ||
| + | |style="text-align:right;"|אח"כ תחלק כ' ורביע שהוא כמות המספרים אשר נקבו להיות להם שרש על אלף ורצ"ו ועולה חלק אחד מס"ד | ||
| + | |- | ||
| + | | | ||
| + | :The cube root of the square root of 1 part of 64 is equal to the thing. | ||
| + | :<math>\scriptstyle{\color{blue}{x=\sqrt[6]{\frac{20+\frac{1}{4}}{36^2}}=\sqrt[6]{\frac{20+\frac{1}{4}}{1296}}=\sqrt[6]{\frac{1}{64}}}}</math> | ||
| + | |style="text-align:right;"|ושרש מעוקב משרש מרובע מא' חלק מס"ד יהיה שוה הדבר | ||
| + | |- | ||
| + | | | ||
| + | :You assumed the first number is 3 things, so multiply 3 by the cube root of the square root of 1 part of 64; the result is a cube root of a square root, or a square root of a cube root of 729 [parts] of 64, which is 1 and a half and this is the first number. | ||
| + | :<math>\scriptstyle{\color{blue}{a=3x=3\sdot\sqrt[6]{\frac{1}{64}}=\sqrt[6]{\frac{729}{64}}=1+\frac{1}{2}}}</math> | ||
| + | |style="text-align:right;"|ואתה הנחת שהמספר הראשון היה ג' דברי‫'<br> | ||
| + | א"כ תכה ג' בשרש מעוקב משרש מרובע מחלק א' מס"ד ועולה שרש מעוקב משרש מרובע או שרש מרובע משרש מעוקב מתשכ"ט מס"ד וזה יבא להיות א' וחצי וככה הוא המספר הראשון | ||
| + | |- | ||
| + | | | ||
| + | :The second number is assumed to be 4 things, so multiply 4 by the cube root of the square root of 1 part of 64; the result is a cube root of a square root, or say: a square root of a cube root of 4[0]96 [parts] of 64, which is 2 and this is the second number. | ||
| + | :<math>\scriptstyle{\color{blue}{b=4x=4\sdot\sqrt[6]{\frac{1}{64}}=\sqrt[6]{\frac{4{\color{red}{0}}96}{64}}=2}}</math> | ||
| + | |style="text-align:right;"|והמספר השני הונח היותו ד' דברים<br> | ||
| + | ולכן תכה ד' בשרש מעוקב משרש מרובע מא' חלק מס"ד ועולה שרש מעוקב משרש מרובע או אמור שרש מרובע משרש מעוקב מתצ"ו מס"ד אשר יבא להיות ב' וככה יבא להיות המספר השני | ||
| + | |- | ||
| + | |Know that this equation has the nature that fits chapter seven, although it exceeds by a square root. | ||
| + | |style="text-align:right;"|ודע כי זאת ההשואה יש לו הטבע הנאות אל הפרק השביעי אע"פ שהולך יותר שרש אחד מרובע | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== Chapter 22 ==== | ||
| + | |||
| + | |style="text-align:right;"|<big>פרק כ"ב</big> | ||
| + | |- | ||
| + | |When numbers are equal to a root of cubes: | ||
| + | :<math>\scriptstyle c=\sqrt{ax^3}</math> | ||
| + | |style="width:45%;text-align:right;"|עוד כאשר המספרי' יהיו שוים אל שרשי המעוקבים | ||
| + | |- | ||
| + | |The numbers should be multiplied by themselves | ||
| + | |style="text-align:right;"|צריך להכות המספרים בעצמם | ||
| + | |- | ||
| + | |Then, this product should be divided by the number of the cubes that are the radicand. | ||
| + | |style="text-align:right;"|ולחלק ההכאה ההיא בכמות המעוקבי' {{#annot:term|1073,2300|AA9e}}הנקובים היות להם שרשים{{#annotend:AA9e}} | ||
| + | |- | ||
| + | |The cube root of the result is equal to the thing. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{x=\sqrt[3]{\frac{c^2}{a}}}}</math> | ||
| + | |style="text-align:right;"|ושרש המעוקב ממה שיעלה יבא לשוות הדבר | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:a/b=⅗;(a√a+b√b)√8=100|618|dp2A}}Find me two numbers such that the one is a part of the other as 3 is of 5; | ||
| + | :and when each is multiplied by its root and both products are summed together, then the sum is multiplied by the root of 8, it yields 100. | ||
| + | :I ask: how much will each number be? | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle a:b=3:5\\\scriptstyle\left[\left(a\sdot\sqrt{a}\right)+\left(b\sdot\sqrt{b}\right)\right]\sdot\sqrt{8}=100\end{cases}</math> | ||
| + | |style="text-align:right;"|תמצא לי שני מספרי' שיהיה חלק האחד מהאחר כמו שהוא ג' מה‫'<br> | ||
| + | וכשיוכה כל אחד על שרשו ויחוברו שתי אלו ההכאות יחד וזה הסך יוכה בשרש ח' יעשה מאה<br> | ||
| + | אשאל כמה יבא להיות כל אחד מהמספרים{{#annotend:dp2A}} | ||
| + | |- | ||
| + | | | ||
| + | :This is its rule: | ||
| + | |style="text-align:right;"|זהו כללו | ||
| + | |- | ||
| + | | | ||
| + | :Suppose the first number is three things [<math>\scriptstyle{\color{blue}{a=3x}}</math>] and the other is five things [<math>\scriptstyle{\color{blue}{b=5x}}</math>] | ||
| + | |style="text-align:right;"|תניח שהמספר הראשון יהיה ג' דברים והאחר ה' דברים | ||
| + | |- | ||
| + | | | ||
| + | :Know that since the root of the thing [<math>\scriptstyle{\color{blue}{\sqrt{x}}}</math>] is inexpressible, the first number should have been defined as three squares [<math>\scriptstyle{\color{blue}{3x^2}}</math>], and the second number as five squares <math>\scriptstyle{\color{blue}{5x^2}}</math>, as the square has a proper root, which is one thing [<math>\scriptstyle{\color{blue}{\sqrt{x^2}=x}}</math>]. | ||
| + | |style="text-align:right;"|<big>דע</big> כי מן הדין היה שבעבור כי שרש הדבר אינו נכר שהיה ראוי שיונח המספר הראשון ג' צינסי והאחר ה' צינסי מפני שהצינסו יש לו היטב שרש שהוא דבר אחד | ||
| + | |- | ||
| + | | | ||
| + | :But, this is the same, since 3 things multiplied by a root of 3 things [<math>\scriptstyle{\color{blue}{3x\sdot\sqrt{3x}}}</math>] is similar to the product of 3 squares by their root [<math>\scriptstyle{\color{blue}{3x^2\sdot\sqrt{3x^2}}}</math>], which is as many things as a root of 3. | ||
| + | |style="text-align:right;"|וכמו זה ישוה מפני כי ככה ישוה ג' דברים מוכים בשרש ג' דברים כמו הכאת ג' צינסו בשרשו אשר יהיה כל כך דברים כמו שהוא שרש ג‫' | ||
| + | |- | ||
| + | | | ||
| + | :Now, multiply the first number, which is 3 things, by its root, which is a root of 3 things; the result is a root of 27 cubes. | ||
| + | :<math>\scriptstyle{\color{blue}{3x\sdot\sqrt{3x}=\sqrt{27x^3}}}</math> | ||
| + | |style="text-align:right;"|עתה תכה המספר ראשון שהוא ג' דברים בשרשם שהוא שרש ג' דברים ועולה שרש כ"ז מעוקבים | ||
| + | |- | ||
| + | | | ||
| + | :Multiply the second number, which is 5 things, by its root; the result is a root of 125 cubes. | ||
| + | :<math>\scriptstyle{\color{blue}{5x\sdot\sqrt{5x}=\sqrt{125x^3}}}</math> | ||
| + | |style="text-align:right;"|עתה תכה המספר השני שהוא ה' דברים בשרשם ועולה שרש קכ"ה מעוקבי‫' | ||
| + | |- | ||
| + | | | ||
| + | :Multiply the sum of a root of 27 cubes plus a root of 125 cubes by the root of 8; the result is a root of 216 cubes plus a root of 1000 cubes and these two roots are equal to 100. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(\sqrt{27x^3}+\sqrt{125x^3}\right)\sdot\sqrt{8}=\sqrt{216x^3}+\sqrt{1000x^3}=100}}</math> | ||
| + | |style="text-align:right;"|שרש כ"ז מעוקבי' ושרש קכ"ה מעוקבי' וזה הסך תכה בשרש ח' ויעלה שרש רי"ו מעוקבים ושרש אלף מעוקבים ואלו שני השרשים הם שוים אל ק‫' | ||
| + | |- | ||
| + | | | ||
| + | :Pursue the above mentioned rule: | ||
| + | |style="text-align:right;"|תרדוף כמו הכלל האמור למעלה | ||
| + | |- | ||
| + | | | ||
| + | :Multiply the numbers that are 100 [by themselves]; the result is 10000. | ||
| + | :<math>\scriptstyle{\color{blue}{100^2=10000}}</math> | ||
| + | |style="text-align:right;"|תכה המספרי' שהם ק' ועולה עשרת אלפים | ||
| + | |- | ||
| + | | | ||
| + | :Divide it by the number of the cubes that are the radicand, i.e. by a root of 216 and a root of 1000: | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt{216}+\sqrt{1000}}}</math> | ||
| + | |style="text-align:right;"|ואלו תחלק על כמות המעוקבים {{#annot:term|1073,2300|5VRC}}הנקובים להיות להם שרש{{#annotend:5VRC}} דהיינו בשרש רי"ו ובשרש אלף | ||
| + | |- | ||
| + | | | ||
| + | :Divide it this way according to the teaching of the division of roots: multiply a root of 1000 plus a root of [216] by a root of 1000 minus a root of 216; the result is 784, which I define as the divisor. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(\sqrt{216}+\sqrt{1000}\right)\sdot\left(\sqrt{1000}-\sqrt{216}\right)=1000-216=784}}</math> | ||
| + | |style="text-align:right;"|אשר זה תחלק באופן זה כפי התלמדות החלוק בשרשים תכה שרש אלף ושרש תשפ"ד בשרש אלף פחות שרש רי"ו אשר עולה תשפ"ד אשר אניח להיות מחלק | ||
| + | |- | ||
| + | | | ||
| + | :<span style=color:Green>'''Rule of three:'''</span> Now say: if 784 yields a root of 1000 minus a root of 216, how much does 1000[0] yield, which is the number multiplied by itself? | ||
| + | :<math>\scriptstyle{\color{blue}{784:\left(\sqrt{1000}-\sqrt{216}\right)=1000{\color{red}{0}}:X}}</math> | ||
| + | |style="text-align:right;"|ועתה אמור כן אם מתשפ"ד יבא שרש אלף פחות שרש רי"ו כמה יבא מאלף שהוא המספר שהוכה בעצמו | ||
| + | |- | ||
| + | | | ||
| + | :Multiply a root of 1000 minus a root of 216 by 1000[0]; the result is a root of 100000000[000] minus a root of 216000000[00]. | ||
| + | |style="text-align:right;"|תכה שרש אלף פחות שרש רי"ו באלף שעולה שרש מ 100000000 פחות שרש מ216000000 | ||
| + | |- | ||
| + | | | ||
| + | :Divide this product by 784; the result is a root of 1626[00] and 569344[00] parts of 614656 minus a root of 351[00] plus 255744[00] parts of 614656. | ||
| + | |style="text-align:right;"|וזאת ההכאה תחלק בתשפ"ד אשר יעלה מזה שרש אלף תרכ"ו וכך חלקים 569344 מ614656 פחות שרש משנ"א וכך חלקים 255744 מ614656 | ||
| + | |- | ||
| + | | | ||
| + | :The cube root of this result is equal to the thing. | ||
| + | |style="text-align:right;"|ושרש מעוקב מזה שעלה יבא לשוות יבא לשוות הדבר | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle x&\scriptstyle=\sqrt[3]{\frac{1000{\color{red}{0}}\sdot\left(\sqrt{1000}-\sqrt{216}\right)}{784}}=\sqrt[3]{\frac{\sqrt{100000000{\color{red}{000}}}-\sqrt{216000000{\color{red}{00}}}}{784}}\\&\scriptstyle=\sqrt[3]{\sqrt{1626{\color{red}{00}}+\frac{569344{\color{red}{00}}}{614656}}-\sqrt{351{\color{red}{00}}+\frac{255744{\color{red}{00}}}{614656}}}\\\end{align}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :You assumed the first number is 3 things, so multiply 3 by a cube root of it: | ||
| + | |style="text-align:right;"|ואתה הנחת שהמספר הראשון יהיה ג' דברי' א"כ תכה ג' בשרש מעוקב מזה | ||
| + | |- | ||
| + | | | ||
| + | :3 should be converted into a square root and this square should be converted into a cube root; you get a [square] root of a cube root of 729. | ||
| + | |style="text-align:right;"|שצריך להשיב ג' אל שרש מרובע ואותו הרבוע צריך להשיב אל שרש מעוקב ויהיה לך שרש משרש מעוקב מתשכ"ט | ||
| + | |- | ||
| + | | | ||
| + | :Multiply a [square] root of a cube root of 729 by a [square] root of a cube root of 1626[00] and 569344[00] parts of 614656 minus a [square] root of a cube root of 351[00] plus 255744[00] parts of 614656; the result is a [square] root of a cube root of 1186029[00] and 158976[00] parts of 614656 minus a root of 256182[00] and 196608[00] parts of 614656 and this is the first number. | ||
| + | |style="text-align:right;"|עתה תכה {{#annot:term|2634|Cbs1}}שרש משרש מעוקב מ{{#annotend:Cbs1}}תשכ"ט בשרש משרש מעוקב מאלף תרכ"ו וחלקי' ה' מאות וס"ט אלפים ושמ"ד מתרי"ד אלפים ותרנ"ו פחות שרש משרש מעוקב משנ"א וחלקים רנ"ה אלפים ותשמ"ד מתרי"ד אלפים ותרנ"ו<br> | ||
| + | ועולה שרש משרש מעוקב מאלף אלפים וקפ"ו אלפים וכ"ט וחלקי' קנ"ח אלפים ותתקע"ו מתרי"ד אלפים ותרנ"ו פחות שרש משרש מעוקב מרנ"ו אלפים מקפ"ב וחלקי' קצ"ו אלפים ותר"ח מתרי"ד אלפים ותרנ"ו וככה יבא להיות המספר הראשון | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle a=3x&\scriptstyle=3\sdot\sqrt[3]{\sqrt{1626{\color{red}{00}}+\frac{569344{\color{red}{00}}}{614656}}-\sqrt{351{\color{red}{00}}+\frac{255744{\color{red}{00}}}{614656}}}\\&\scriptstyle=\sqrt[3]{\sqrt{729}}\sdot\sqrt[3]{\sqrt{1626{\color{red}{00}}+\frac{569344{\color{red}{00}}}{614656}}-\sqrt{351{\color{red}{00}}+\frac{255744{\color{red}{00}}}{614656}}}\\&\scriptstyle=\sqrt[3]{\sqrt{1186029{\color{red}{00}}+\frac{158976{\color{red}{00}}}{614656}}-\sqrt{256182{\color{red}{00}}+\frac{196608{\color{red}{00}}}{614656}}}\\\end{align}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :You assumed the second number is 5 things, so multiply 5 by the thing: | ||
| + | |style="text-align:right;"|ואתה הנחת שהמספר השני היותו ה' דברי' א"כ תכה ה' במה ששוה הדבר | ||
| + | |- | ||
| + | | | ||
| + | :Convert 5 into a [square] of a cube root; you get a [square] root of a cube root of 15625. | ||
| + | |style="text-align:right;"|תשיב ה' אל שרש משרש מעוקב ויהיה לך שרש משרש מעו' מט"ו אלפים ותרכ"ה | ||
| + | |- | ||
| + | | | ||
| + | :Now, multiply a [square] root of a cube root of 15625 by a [square] root of a cube root of 1626[00] and 569344[00] parts of 614656 minus a [square] root of a cube root of 351[00] plus 255744[00] parts of 614656; the result is a [square] root of a cube root of 25420723[00] and 83712[00] parts of 614656 minus a root of 5490876[00] and 121344[00] parts of 614656 and this is the second number. | ||
| + | |style="text-align:right;"|עתה תכה {{#annot:term|2634|Ihbw}}שרש משרש מעו' מ{{#annotend:Ihbw}}ט"ו אלפים ותרכ"ה בשרש משרש מעו' מאלף ותרכ"ו וחלקי' מתקס"ט אלפים ושמ"ד מתרי"ד אלפים ותרנ"ו פחות שרש משרש מעו' משנ"א וחלקים רנ"ה אלפים ותשמ"ד מתרי"ד אלפים ותרנ"ו<br> | ||
| + | ויעלה שרש משרש מעו' מכ"ה אלפי אלפים ות"כ אלפי' ותשכ"ג וחלקי' פ"ג אלפי' ותשי"ב מתרי"ד אלפי' ותרנ"ו פחות שרש מעו' משרש מה' אלפי אלפים ות"צ אלפים ותתע"ו וחלקי' קכ"א אלפים ושמ"ד מן תרי"ד אלפים ותרנ"ו<br> | ||
| + | וככה יבא להיות המספר השני | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle b=5x&\scriptstyle=5\sdot\sqrt[3]{\sqrt{1626{\color{red}{00}}+\frac{569344{\color{red}{00}}}{614656}}-\sqrt{351{\color{red}{00}}+\frac{255744{\color{red}{00}}}{614656}}}\\&\scriptstyle=\sqrt[3]{\sqrt{15625}}\sdot\sqrt[3]{\sqrt{1626{\color{red}{00}}+\frac{569344{\color{red}{00}}}{614656}}-\sqrt{351{\color{red}{00}}+\frac{255744{\color{red}{00}}}{614656}}}\\&\scriptstyle=\sqrt[3]{\sqrt{25420723{\color{red}{00}}+\frac{83712{\color{red}{00}}}{614656}}-\sqrt{5490876{\color{red}{00}}+\frac{121344{\color{red}{00}}}{614656}}}\\\end{align}}}</math> | ||
| + | |- | ||
| + | |Know that this equation is of the nature of chapter 7, even though it yields an extra root, since these two roots, i.e. the products received above, cannot be combined together to one root alone by their expression or their sum. | ||
| + | |style="text-align:right;"|ודע כי זאת ההשואה היא מטבע הפרק השביעי אע"פ שתענה שרש אחד יותר מפני כי אותם שני שרשי' דהיינו ההכאות שנעשו למעלה אי אפשר לחברם יחד בענותם או בחברם בשרש אחד לבד | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== Chapter 23 ==== | ||
| + | |||
| + | |style="width:45%;text-align:right;"|<big>פרק כ"ג</big> | ||
| + | |- | ||
| + | |When the squares of squares are equal to a root of a number: | ||
| + | :<math>\scriptstyle ax^4=\sqrt{c}</math> | ||
| + | |style="text-align:right;"|כאשר הצינסי מצינסי יהיו שוים אל שרשי מספרי‫' | ||
| + | |- | ||
| + | |The number of the squares of squares should be multiplied by itself. | ||
| + | |style="text-align:right;"|צריך להכות כמות הצינסי מצינסי בעצמם | ||
| + | |- | ||
| + | |Then, the number that is the radicand sould be divided by this product. | ||
| + | |style="text-align:right;"|ולחלוק המספרי' הנקובי' על זאת ההכאה | ||
| + | |- | ||
| + | |Extract the root of the root of the root of the result and this is equal to the thing. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{x=\sqrt[8]{\frac{c}{a^2}}}}</math> | ||
| + | |style="text-align:right;"|ו{{#annot:term|795|QuYq}}תקח מהעולה שרש השרש מהשרש{{#annotend:QuYq}} וככה יבא לשוות הדבר | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:(a·⅔a)²=√50|618|MvD8}}Find me a number, such that when it is multiplied by its two thirds, and that product is multiplied by itself, it yields the root of fifty. | ||
| + | :I ask: how much will the number be? | ||
| + | :<math>\scriptstyle\left(a\sdot\frac{2}{3}a\right)^2=\sqrt{50}</math> | ||
| + | |style="text-align:right;"|עשה לי זה החשבון תמצא לי מספר אחד שכאשר הוכה בשני שלישיו ואותה ההכאה תוכה בעצמה תעשה שרש חמשים<br> | ||
| + | אשאל כמה יבא להיות המספר האמור{{#annotend:MvD8}} | ||
| + | |- | ||
| + | | | ||
| + | :This is its rule: | ||
| + | |style="text-align:right;"|זהו כללו | ||
| + | |- | ||
| + | | | ||
| + | :Suppose the number is one thing [<math>\scriptstyle{\color{blue}{a=x}}</math>]. | ||
| + | |style="text-align:right;"|תניח שהמספר יהיה דבר אחד | ||
| + | |- | ||
| + | | | ||
| + | :One thing is multiplied by its two-thirds; the result is 2-thirds of a square. | ||
| + | |style="text-align:right;"|ויוכה דבר אחד בשני שלישיו ועולה ב' שלישי' מצינסו | ||
| + | |- | ||
| + | | | ||
| + | :Now, multiply 2-thirds of a square by themselves; the result is 4-ninths of a square of a square that are equal to a root of 50. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(x\sdot\frac{2}{3}x\right)^2=\left(\frac{2}{3}x^2\right)^2=\frac{4}{9}x^4=\sqrt{50}}}</math> | ||
| + | |style="text-align:right;"|עתה תכה ב' שלישי' מצינסו בעצמו ועולה ד' תשיעיות מצינסו מצינסו שהם שוים אל שרש נ‫' | ||
| + | |- | ||
| + | | | ||
| + | :Pursue the above mentioned rule: | ||
| + | |style="text-align:right;"|עתה תרדוף כפי הכלל האמור למעלה | ||
| + | |- | ||
| + | | | ||
| + | :Multiply the number of the squares of the squares, which is 4-ninths, by itself; the result is 16 parts of 81. | ||
| + | |style="text-align:right;"|תכה כמות הצינסי מצינסי בעצמם שהם ד' תשיעיות ויעלה י"ו חלקים מפ"א | ||
| + | |- | ||
| + | | | ||
| + | :Divide the number that is the radicand [by it]; the result is 253 and an eighth. | ||
| + | |style="text-align:right;"|ותחלק המספרי' הנקובי' היות להם שרש ויעלה מזה רנ"ג ושמינית | ||
| + | |- | ||
| + | | | ||
| + | :The root of the root of its root is the thing and so is the said number. | ||
| + | :<math>\scriptstyle{\color{blue}{x=\sqrt[8]{\frac{50}{\left(\frac{4}{9}\right)^2}}=\sqrt[8]{\frac{50}{\frac{16}{81}}}=\sqrt[8]{253+\frac{1}{8}}}}</math> | ||
| + | |style="text-align:right;"|ושרש השרש משרשו יבא לשוות הדבר וככה יבא להיות המספר האמור | ||
| + | |- | ||
| + | |Know that this is almost the same as the nature of chapter 11, except that here a root of one degree higher is extracted. | ||
| + | |style="text-align:right;"|ודע כי זה הוא כמעט דומה לטבע הפרק הי"א מלבד שלוקח שרש אחד יותר | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== Chapter 24 ==== | ||
| + | |||
| + | |style="width:45%;text-align:right;"|<big>פרק כ"ד</big> | ||
| + | |- | ||
| + | |When the numbers are equal to a root of the squares of squares: | ||
| + | :<math>\scriptstyle c=\sqrt{ax^4}</math> | ||
| + | |style="text-align:right;"|כאשר המספרים הם שוים אל שרשי הסינסי מצינסי | ||
| + | |- | ||
| + | |The numbers should be multiplied by themselves. | ||
| + | |style="text-align:right;"|צריך להכות המספרי' בעצמם | ||
| + | |- | ||
| + | |Then, this product should be divided by the number of the squares of squares that are the radicand. | ||
| + | |style="text-align:right;"|ולחלק אותה ההכאה בכמות הצינסי מצינסי הנקובי' היות להם שרש | ||
| + | |- | ||
| + | |The root of the root of the result should be extracted and this is equal to the thing. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{x=\sqrt[4]{\frac{c^2}{a}}}}</math> | ||
| + | |style="text-align:right;"|ולקחת מהעולה שרש שרשו וככה יבא לשוות הדבר | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:a/b=⅔;ab√8=100|618|mV9d}}Find me two numbers such that one is a part of the other as 2 is of 3; | ||
| + | :and when the one is multiplied by the other, and the product is multiplied by the root of 8, it yields 100. | ||
| + | :I ask: how much will each number be? | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle a:b=2:3\\\scriptstyle\left(a\sdot b\right)\sdot\sqrt{8}=100\end{cases}</math> | ||
| + | |style="text-align:right;"|תמצא לי שני מספרי' שיהיה חלק האחד מהאחר כמו שב' הוא מג‫'<br> | ||
| + | וכשיוכה האחד באחר ואותה ההכאה תוכה בשרש ח' ועושה ק‫'<br> | ||
| + | אשאל כמה יבא להיות כל אחד מהמספרי‫'{{#annotend:mV9d}} | ||
| + | |- | ||
| + | | | ||
| + | :This is its rule: | ||
| + | |style="text-align:right;"|זהו כללו | ||
| + | |- | ||
| + | | | ||
| + | :Suppose the first number is two things [<math>\scriptstyle{\color{blue}{a=2x}}</math>] and the other must be three things [<math>\scriptstyle{\color{blue}{b=3x}}</math>] | ||
| + | |style="text-align:right;"|תניח שהמספר ראשון ב' דברים והאחר מחוייב שיהיה ג' דברים | ||
| + | |- | ||
| + | | | ||
| + | :Now, multiply the first by the second, which is 2 things by 3 things; the result is 6 squares. | ||
| + | |style="text-align:right;"|עתה תכה הראשון בשני שהוא ב' דברי' על ג' דברים ועולה ו' צינסי | ||
| + | |- | ||
| + | | | ||
| + | :Multiply 6 squares by a root of 8: | ||
| + | |style="text-align:right;"|תכה ו' צינסי על שרש ח‫' | ||
| + | |- | ||
| + | | | ||
| + | :Know that you have to convert the 6 squares into roots; when they are converted into roots, they are a root of 36 squares of squares. | ||
| + | |style="text-align:right;"|דע כי הנך צריך להשיב ו' צינסי אל שרשים והם בשהושבו אל שרשים יהיו שרש מל"ו צינסי מצינסי | ||
| + | |- | ||
| + | | | ||
| + | :Multiply the root of 36 squares of squares by a root of 8; the result is a root of 288 squares of squares that is equal to 100 numbers. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(2x\sdot3x\right)\sdot\sqrt{8}=6x^2\sdot\sqrt{8}=\sqrt{36x^4\sdot8}=\sqrt{288x^4}=100}}</math> | ||
| + | |style="text-align:right;"|עתה תכה שרש מל"ו צינסי מצינסי בשרש ח' עולה שרש מרפ"ח צינסי מצינסי שהם שוים אל ק' מספרי‫' | ||
| + | |- | ||
| + | | | ||
| + | :Pursue the above mentioned rule: | ||
| + | |style="text-align:right;"|עתה תרדוף כפי הכלל האמור למעלה | ||
| + | |- | ||
| + | | | ||
| + | :Multiply the numbers that are 100 by themselves; the result is 10000. | ||
| + | |style="text-align:right;"|תכה המספרי' בעצמם שהם ק' ועולה עשרת אלפים | ||
| + | |- | ||
| + | | | ||
| + | :Divide it by the number of the squares of squares that are the radicand, which is 288; the result is 34 and 13 parts of 18 and this is equal to the thing. | ||
| + | :<math>\scriptstyle{\color{blue}{x=\sqrt[4]{\frac{100^2}{288}}=\sqrt[4]{\frac{10000}{288}}=\sqrt[4]{34+\frac{13}{18}}}}</math> | ||
| + | |style="text-align:right;"|ואלו תחלק על כמות הצינסי מצינסי הנקובים היות להם שרשים והם רפ"ח שעולה מזה ל"ד וי"ג חלקי' מי"ח וככה יבא לשוות הדבר | ||
| + | |- | ||
| + | | | ||
| + | :You assumed the first number is 2 things, so multiply 2 by the value of the thing: | ||
| + | |style="text-align:right;"|ואתה הנחת שהמספר ראשון היה ב' דברי‫'<br> | ||
| + | א"כ תכה ב' על מה ששוה הדבר | ||
| + | |- | ||
| + | | | ||
| + | :Convert 2 into a root of a root; the result is 16. | ||
| + | |style="text-align:right;"|תשיב ב' אל שרש משרש שיבא להיות י"ו | ||
| + | |- | ||
| + | | | ||
| + | :Now, multiply a root of a root of 16 by a root of a root of 34 and 13 parts of 18; the result is a root of a root of 555 [and 10 parts of 18] and this is the first number. | ||
| + | :<math>\scriptstyle{\color{blue}{a=2x=2\sdot\sqrt[4]{34+\frac{13}{18}}=\sqrt[4]{16\sdot\left(34+\frac{13}{18}\right)}=\sqrt[4]{555+{\color{red}{\frac{10}{18}}}}}}</math> | ||
| + | |style="text-align:right;"|עתה תכה שרש משרש מי"ו בשרש שרש ל"ד וי"ג חלקים מי"ח ועולה שרש שרש מתקנ"ה וככה הוא המספר הראשון | ||
| + | |- | ||
| + | | | ||
| + | :You assumed the second is 3 things, so multiply [3] by a root of a root of 34 and 13 parts of 18; the result is a root of a root of 2812 and a half and this is the second number. | ||
| + | :<math>\scriptstyle{\color{blue}{b=3x=3\sdot\sqrt[4]{34+\frac{13}{18}}=\sqrt[4]{2812+\frac{1}{2}}}}</math> | ||
| + | |style="text-align:right;"|והשני הנחת היותו ג' דברים<br> | ||
| + | א"כ תכה שרש משרש ל"ד וי"ג חלקים מי"ח ועולה שרש משרש מאלפים ותתי"ב וחצי וככה יבא להיות המספר השני | ||
| + | |- | ||
| + | |Know that this equation can be returned to chapter 11. | ||
| + | |style="text-align:right;"|ודע כי זאת ההשואה אפש' להשיבה אל הפרק הי"א | ||
| + | |- | ||
| + | |If the number of the squares of squares that are the radicand has a root, it can be returned to the second chapter. | ||
| + | |style="text-align:right;"|ואם לכמות הצינסי מהצינס' הנקובים להיות להם שרש היה להם שרש היתה מושבת אל הפרק השני | ||
| + | |- | ||
| + | |Because its root is a square. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\sqrt{x^4}=x^2}}</math> | ||
| + | |style="text-align:right;"|מפני כי שרשו היה צינסו | ||
| + | |- | ||
| + | |So, it becomes equal to the numbers. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{c=\sqrt{ax^4}\;\longrightarrow\;c=\sqrt{a}x^2}}</math> | ||
| + | |style="text-align:right;"|ויבא להיות שוה אל המספרי‫' | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== Chapter 25 ==== | ||
| + | |||
| + | |style="width:45%;text-align:right;"|<big>פרק כ"ה</big> | ||
| + | |- | ||
| + | |When things are equal to a root of things: | ||
| + | :<math>\scriptstyle ax=\sqrt{bx}</math> | ||
| + | |style="text-align:right;"|כאשר הדברים הם שוים אל שרשי הדברים | ||
| + | |- | ||
| + | |The [number] of the things should be multiplied by itself and this product is the divisor. | ||
| + | |style="text-align:right;"|צריך להכות שרשי הדברים בעצמם ואותה ההכאה {{#annot:term|459,1487|osZk}}תחזיק ל{{#annotend:osZk}}מחלק | ||
| + | |- | ||
| + | |Divide [the number of] the things that are the radicand by the said product, i.e. by the square of the number of the things; the result is equal to the thing. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{x=\frac{b}{a^2}}}</math> | ||
| + | |style="text-align:right;"|והדברי' אשר נקבו תחלק בהכאה האמורה דהיינו ברבוע כמות הדברי‫'<br> | ||
| + | ומה שיעלה ככה מספרי' יבא לשוות הדבר | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:a/b=¾;8a=6√b|618|8HYy}}Find me two numbers such that the one is a part of the second as 3 is of 4; | ||
| + | :and the first multiplied by 8, yields the same as the root of the second multiplied by 6. | ||
| + | :I ask: how much will each number be? | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle a:b=3:4\\\scriptstyle8a=6\sqrt{b}\end{cases}</math> | ||
| + | |style="text-align:right;"|תמצא לי שני מספרי' שיהיה החלק האחד מהשני כמו שהוא ג' מד‫'<br> | ||
| + | וכן יעשה הראשון מוכה בח' כמו השני דהיינו שרשו מוכה בו‫'<br> | ||
| + | אשאל כמה יבא להיות כל אחד מהמספרים{{#annotend:8HYy}} | ||
| + | |- | ||
| + | | | ||
| + | :This is its rule: | ||
| + | |style="text-align:right;"|זהו כללו | ||
| + | |- | ||
| + | | | ||
| + | :Suppose the first is three things [<math>\scriptstyle{\color{blue}{a=3x}}</math>] and the other is four things [<math>\scriptstyle{\color{blue}{b=4x}}</math>]. | ||
| + | |style="text-align:right;"|תניח שהראשון יהיה ג' דברי' והאחר ד' דברים | ||
| + | |- | ||
| + | | | ||
| + | :Now, multiply the first, which is 3 things, by 8; the result is 24 things. | ||
| + | :<math>\scriptstyle{\color{blue}{8\sdot3x=24x}}</math> | ||
| + | |style="text-align:right;"|עתה תכה הראשון שהוא ג' דברי' בח' ועולה כ"ד דברי‫' | ||
| + | |- | ||
| + | | | ||
| + | :Then, multiply the second, which is a root of 4 things, by 6; the result is a root of 144 squares and it is equal to 24 things. | ||
| + | :<math>\scriptstyle{\color{blue}{6\sdot\sqrt{4x}=\sqrt{144x^2}=24x}}</math> | ||
| + | |style="text-align:right;"|אח"כ תכה שרש השני שהוא שרש ד' דברים בו' ועולה שרש קמ"ד צינסי והוא שוה אל כ"ד דברי‫' | ||
| + | |- | ||
| + | | | ||
| + | :Pursue the above mentioned rule: | ||
| + | |style="text-align:right;"|עתה תרדוף כפי הכלל האמור למעלה | ||
| + | |- | ||
| + | | | ||
| + | :Multiply the number of the things, which is 24, by itself; the result is 576. | ||
| + | |style="text-align:right;"|תכה כמות הדברי' בעצמם שהוא כ"ד ועולה תקע"ו | ||
| + | |- | ||
| + | | | ||
| + | :Divide the number of the things that are the radicand, which is 144, by 576; the result is a quarter and this is equal to the thing. | ||
| + | :<math>\scriptstyle{\color{blue}{x=\frac{144}{24^2}=\frac{144}{576}=\frac{1}{4}}}</math> | ||
| + | |style="text-align:right;"|ותחלק הדברי' הנקובי' היות להם שרשי' והם קמ"ד על תקע"ו ועולה מזה א' רביע וזה יבא לשוות הדבר | ||
| + | |- | ||
| + | | | ||
| + | :You assumed the first number is 3 things, so multiply 3 by the said quarter; the result is 3-quarters of a number, i.e. the 3 things you have assumed to be the first number, and this is the first number. | ||
| + | :<math>\scriptstyle{\color{blue}{a=3x=3\sdot\frac{1}{4}=\frac{3}{4}}}</math> | ||
| + | |style="text-align:right;"|ואתה הנחת המספר ראשון היותו ג' דברים<br> | ||
| + | א"כ תכה ג' בא' רביע האמור ועולה ג' רביעי' ממספר דהיינו הג' דברי' אשר הנחת אותם בעד המספר הראשון וככה יבא להיות המספר הראשון | ||
| + | |- | ||
| + | | | ||
| + | :You assumed the second number is 4 things, so multiply the said quarter by 4; the result is 4-quarters of a number, which is one integer, and this is the second number. | ||
| + | :<math>\scriptstyle{\color{blue}{b=4x=4\sdot\frac{1}{4}=\frac{4}{4}=1}}</math> | ||
| + | |style="text-align:right;"|ואתה הנחת היות המספר השני ד' דברים<br> | ||
| + | א"כ תכה הרביע האמור בד' ועולה ד' רביעים ממספר והם אחד שלם וככה יבא להיות המספר השני | ||
| + | |- | ||
| + | |Know that this equation can be returned to the third chapter: | ||
| + | |style="text-align:right;"|ודע כי זאת ההשואה אפש' להשיבה אל הפרק השלישי | ||
| + | |- | ||
| + | |Since a root of a thing multiplied by a root of a thing yields a thing. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\sqrt{x}\sdot\sqrt{x}=x}}</math> | ||
| + | |style="text-align:right;"|מפני כי שרש דבר מוכה בשרש דבר עושה דבר | ||
| + | |- | ||
| + | |A thing multiplied by a thing yields a square. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{x\sdot x=x^2}}</math> | ||
| + | |style="text-align:right;"|ודבר מוכה בדבר עושה צינסו | ||
| + | |- | ||
| + | |Hence, by multiplying each part [of the equation] by itself: | ||
| + | |style="text-align:right;"|א"כ בהכות כל אחד מהחלקים בעצמו | ||
| + | |- | ||
| + | |The root of the things becomes things. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\left(\sqrt{bx}\right)^2=bx}}</math> | ||
| + | |style="text-align:right;"|שרשי הדברים יבאו דברים | ||
| + | |- | ||
| + | |The things become squares. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\left(ax\right)^2=Ax^2}}</math> | ||
| + | |style="text-align:right;"|והדברים יבאו צינסו | ||
| + | |- | ||
| + | |Then, by dividing the whole equation by the things, the aforesaid calculation is returned to the first chapter. | ||
| + | |style="text-align:right;"|ובחלק אחר זה כל ההשואה על הדברי' ישוב החשבון האמור למעלה אל הפרק הראשון | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== Chapter 26 ==== | ||
| + | |||
| + | |style="width:45%;text-align:right;"|<big>פרק כ"ו</big> | ||
| + | |- | ||
| + | |When the squares are equal to a root of things: | ||
| + | :<math>\scriptstyle ax^2=\sqrt{bx}</math> | ||
| + | |style="text-align:right;"|עוד כאשר הצינסו הם שוים אל שרשי דברים | ||
| + | |- | ||
| + | |The number of the squares should be multiplied by itself. | ||
| + | |style="text-align:right;"|צריך להכות כמות הצינסי בעצמם | ||
| + | |- | ||
| + | |Then, the number of the things that are the radicand should be divided by this product, or by the square of [the number of] the squares. | ||
| + | |style="text-align:right;"|ולחלק כמות הדברי' הנקובי' להיות להם שרשים על אותה ההכאה או רבוע הצינסי | ||
| + | |- | ||
| + | |Its cube root is equal to the thing. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{x=\sqrt[3]{\frac{b}{a^2}}}}</math> | ||
| + | |style="text-align:right;"|ושרשו המעוקב יבא לשוות הדבר | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:a/b=⅖;ab=8√b|618|BstD}}Find me two numbers such that the one is a part of the other as 2 is of 5; | ||
| + | :and when the first is multiplied by the second, it yields the same as the product of the root of the second by 8. | ||
| + | :I ask: how much will each number be? | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle a:b=2:5\\\scriptstyle a\sdot b=8\sqrt{b}\end{cases}</math> | ||
| + | |style="text-align:right;"|תמצא לי שני מספרי' שיהיה האחד חלק מהאחר כמו שב' הוא מה‫'<br> | ||
| + | וכשהוכה האחד בשני יעשה כמו הכאת שרש השני בח‫'<br> | ||
| + | אשאל כמה יבא להיות כל אחד מהמספרי‫'{{#annotend:BstD}} | ||
| + | |- | ||
| + | | | ||
| + | :This is its rule: | ||
| + | |style="text-align:right;"|זהו כללו | ||
| + | |- | ||
| + | | | ||
| + | :Suppose the first number is two things [<math>\scriptstyle{\color{blue}{a=2x}}</math>] and the other is five things [<math>\scriptstyle{\color{blue}{b=5x}}</math>] | ||
| + | |style="text-align:right;"|תניח שהמספר הראשון יהיה ב' דברי' והאחר יהיה ה' דברי‫' | ||
| + | |- | ||
| + | | | ||
| + | :Now, multiply the first by the second, which is 2 things by 5 things; the result is ten squares. Keep them for one side of the equation. | ||
| + | :<math>\scriptstyle{\color{blue}{2x\sdot5x=10x^2}}</math> | ||
| + | |style="text-align:right;"|עתה תכה הראשון בשני שהוא ב' דברי' על ה' דברי' ועולה עשרה צינסי ושמרם בעד חלק אחד מההשואה | ||
| + | |- | ||
| + | | | ||
| + | :Multiply the root of the second, which is a root of 5, by 8; the result is a root of 320 things that are equal to ten squares. | ||
| + | :<math>\scriptstyle{\color{blue}{8\sdot\sqrt{5x}=\sqrt{320x}=10x^2}}</math> | ||
| + | |style="text-align:right;"|עתה תכה שרש השני שהוא שרש ה' בח' ועולה שרש מש"כ דברי' שהם שוים אל עשרה צינסי | ||
| + | |- | ||
| + | | | ||
| + | :Pursue the above mentioned rule: | ||
| + | |style="text-align:right;"|עתה תרדוף כפי הכלל האמור למעלה | ||
| + | |- | ||
| + | | | ||
| + | :Multiply the number of the square, which is ten, by itself; the result is ten. | ||
| + | |style="text-align:right;"|תכה כמות הצינסי בעצמם שהם עשרה ויעלה ק‫' | ||
| + | |- | ||
| + | | | ||
| + | :Now, divide the number of the things that are the radicand, which is 320, by 100; the result is 3 and a fifth. | ||
| + | |style="text-align:right;"|עתה תחלק כמות הדברי' אשר נקבו היות להם שרשים והם ש"כ על ק' ויעלה מזה ג' וחומש | ||
| + | |- | ||
| + | | | ||
| + | :The cube root of 3 and a fifth is the thing. | ||
| + | :<math>\scriptstyle{\color{blue}{x=\sqrt[3]{\frac{320}{10^2}}=\sqrt[3]{\frac{320}{100}}=\sqrt[3]{3+\frac{1}{5}}}}</math> | ||
| + | |style="text-align:right;"|ושרש המעוקב מג' וא' חומש יבא להיות הדבר | ||
| + | |- | ||
| + | | | ||
| + | :You assumed the first number is 2 things, so multiply 2 by a cube root of 3 and a fifth; the result is cube root of 25 and 3-fifths and this is the first number. | ||
| + | :<math>\scriptstyle{\color{blue}{a=2x=2\sdot\sqrt[3]{3+\frac{1}{5}}=\sqrt[3]{25+\frac{3}{5}}}}</math> | ||
| + | |style="text-align:right;"|ואתה הנחת שהמספר הראשון היה ב' דברים<br> | ||
| + | א"כ תכה ב' על שרש מעוקב מג' וא' חומש ויעלה שרש מעו' מכ"ה וג' חומשי' וככה יבא להיות המספר ראשון | ||
| + | |- | ||
| + | | | ||
| + | :You assumed the second is 5 things, so multiply 5 by a cube root of 3 and a fifth; the result is cube root of 400 and this is the second number. | ||
| + | :<math>\scriptstyle{\color{blue}{b=5x=5\sdot\sqrt[3]{3+\frac{1}{5}}=\sqrt[3]{400}}}</math> | ||
| + | |style="text-align:right;"|והשני הנחת היותו ה' דברים<br> | ||
| + | א"כ תכה ה' בשרש מעו' מג' וא' חומש ויעלה שרש מעו' מת' וככה יבא להיות המספר השני | ||
| + | |- | ||
| + | |Know that this equation can be returned to chapter 12, when each side [of the equation] is multiplied by itself. | ||
| + | |style="text-align:right;"|ודע כי זאת ההשואה אפש' להשיבה אל הפרק הי"ב כאשר יוכה כל אחד מהחלקי' בעצמו | ||
| + | |- | ||
| + | |The result is things equal squares of squares. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\left(ax^2\right)^2=\left(\sqrt{bx}\right)^2\;\longrightarrow\;Ax^4=bx}}</math> | ||
| + | |style="text-align:right;"|והיה בא דברי' שוים אל צינסי מצינסי | ||
| + | |- | ||
| + | |After it is returned to the chapter 12, it can be returned to the seventh chapter, by dividing each side [of the equation] by a thing. | ||
| + | |style="text-align:right;"|ועוד אפש' להשיבה אל הפרק השביעי אחר שהושבה אל הי"ב בחלק כל אחד מהחלקי' על דבר | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== Chapter 27 ==== | ||
| + | |||
| + | |style="width:45%;text-align:right;"|<big>פרק כ"ז</big> | ||
| + | |- | ||
| + | |When the things are equal to a root of cubes: | ||
| + | :<math>\scriptstyle bx=\sqrt{ax^3}</math> | ||
| + | |style="text-align:right;"|כאשר הדברים יהיו שוים אל שרשי מעוקבים | ||
| + | |- | ||
| + | |The number of the things should be multiplied by itself. | ||
| + | |style="text-align:right;"|צריך להכות כמות הדברים בעצמם | ||
| + | |- | ||
| + | |Then, this product should be divided by the number of the cubes that are the radicand and the result is equal to the thing. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{x=\frac{b^2}{a}}}</math> | ||
| + | |style="text-align:right;"|ולחלק ההכאה ההיא על כמות המעוקבים הנקובי' היות להם שרש והעולה מזה ככה שוה הדבר | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:a/b=⅔;a√a=2b|618|Jb23}}Find me two numbers such that the first is a part of the second as 2 is of 3; | ||
| + | :and when the first is multiplied by its root it yields the same as the product of the second by 2. | ||
| + | :I ask: how much will each number be? | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle a:b=2:3\\\scriptstyle a\sdot\sqrt{a}=2b\end{cases}</math> | ||
| + | |style="text-align:right;"|תמצא לי שני מספרים שיהיה הראשון חלק מהשני כמו שב' הוא מג‫'<br> | ||
| + | וכשיוכה הראשון בשרש עצמו יעשה כמו הכאת השני בשנים<br> | ||
| + | אשאל כמה יבא להיות כל אחד מהמספרים{{#annotend:Jb23}} | ||
| + | |- | ||
| + | | | ||
| + | :This is its rule: | ||
| + | |style="text-align:right;"|זהו כללו | ||
| + | |- | ||
| + | | | ||
| + | :Suppose the first number is two things [<math>\scriptstyle{\color{blue}{a=2x}}</math>] and the second is three things [<math>\scriptstyle{\color{blue}{b=3x}}</math>] | ||
| + | |style="text-align:right;"|תניח השני שהמספר הראשון יהיה ב' דברי' והשני ג' דברי‫' | ||
| + | |- | ||
| + | | | ||
| + | :Now, multiply the first, which is 2 things, by its own root this way: | ||
| + | |style="text-align:right;"|עתה תכה הראשון שהוא ב' דברים בשרש עצמו בזה האופן | ||
| + | |- | ||
| + | | | ||
| + | :Convert 2 things into a square root; you get a root of 4 squares. | ||
| + | |style="text-align:right;"|תשיב ב' דברי' אל שרש מרובע ויהיה לך שרש מד' צינסי | ||
| + | |- | ||
| + | | | ||
| + | :Multiply it by a root of 2 things, i.e. by a root of the first number; the result is a root of 8 cubes. Keep it for one side of the equation. | ||
| + | :<math>\scriptstyle{\color{blue}{2x\sdot\sqrt{2x}=\sqrt{4x^2\sdot2x}=\sqrt{8x^3}}}</math> | ||
| + | |style="text-align:right;"|אשר תכם בשרש ב' דברים דהיינו בשרש המספר הראשון ויעלה שרש ח' מעוקבים ושמרם בעד חלק מההשואה | ||
| + | |- | ||
| + | | | ||
| + | :Now, multiply the other number, which is 3 things, by 2; the result is 6 things, which is the other side. | ||
| + | :<math>\scriptstyle{\color{blue}{2\sdot3x=6x}}</math> | ||
| + | |style="text-align:right;"|עתה תכה המספר האחר שהוא ג' דברים בב' ועולה ו' דברים שהם החלק האחר | ||
| + | |- | ||
| + | | | ||
| + | :You get a root of 8 cubes equals 6 things. | ||
| + | :<math>\scriptstyle{\color{blue}{6x=\sqrt{8x^3}}}</math> | ||
| + | |style="text-align:right;"|ויהיה לך שרש ח' מעוק' שוה לו' דברים | ||
| + | |- | ||
| + | | | ||
| + | :Pursue the above said rule: | ||
| + | |style="text-align:right;"|עתה תרדוף כפי הכלל האמור למעלה | ||
| + | |- | ||
| + | | | ||
| + | :Multiply the number of the things, which is 6, by itself; the result is 36. | ||
| + | |style="text-align:right;"|תכה כמות הדברי' בעצמם והם ו' ועולה ל"ו | ||
| + | |- | ||
| + | | | ||
| + | :Divide the 36 by the number of the cubes that are the radicand, i.e. by 8; the result is 4 and a half and this is equal to the thing. | ||
| + | :<math>\scriptstyle{\color{blue}{x=\frac{6^2}{8}=\frac{36}{8}=4+\frac{1}{2}}}</math> | ||
| + | |style="text-align:right;"|ואלו הל"ו תחלק על כמות המעוקבי' הנקובי' להיות להם שרשים דהיינו על ח' ויבא ד' וחצי וככה שוה הדבר | ||
| + | |- | ||
| + | | | ||
| + | :You assumed the first number is 2 things, so multiply 2 things by 4 and a half; the result is 9 and this is the first number. | ||
| + | :<math>\scriptstyle{\color{blue}{a=2x=2\sdot\left(4+\frac{1}{2}\right)=9}}</math> | ||
| + | |style="text-align:right;"|ואתה הנחת היות המספר הראשון ב' דברי‫'<br> | ||
| + | א"כ תכה ב' דברי' בד' וחצי ועולה ט' וככה שוה המספר הראשון | ||
| + | |- | ||
| + | | | ||
| + | :You assumed the second number is 3 things, so multiply 3 by 4 and a half; the result is 13 and a half and this is the second number. | ||
| + | :<math>\scriptstyle{\color{blue}{b=3x=3\sdot\left(4+\frac{1}{2}\right)=13+\frac{1}{2}}}</math> | ||
| + | |style="text-align:right;"|והשני הנחת היותו ג' דברי‫'<br> | ||
| + | א"כ תכה ג' בד' וחצי ועולה י"ג וחצי וככה הוא המספר השני | ||
| + | |- | ||
| + | |Know that this equation can be returned to the ninth chapter, by multiplying each side of the equation by itself. | ||
| + | |style="text-align:right;"|ודע שזאת ההשואה אפש' להשיבו אל הפרק התשיעי בהכות כל אחד מהחלקי' מההשואות בעצמו | ||
| + | |- | ||
| + | |After it is returned to the ninth chapter, it can then be returned to the first chapter by dividing each side [of the equation] by a square. | ||
| + | |style="text-align:right;"|ובהיותו מושב אל הפרק התשיעי אפשר להשיבה אל הפרק הראשון בחלק כל חלק על צינסי | ||
| + | |- | ||
| + | |By dividing the said equation by a thing, it is returned to the third chapter in the aforesaid way. | ||
| + | |style="text-align:right;"|ובחלק ההשואה האמורה על דברים תשוב אל הפרק השלישי באופן האמור | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== Chapter 28 ==== | ||
| + | |||
| + | |style="width:45%;text-align:right;"|<big>פרק כ"ח</big> | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|עוד באופן אחר | ||
| + | |- | ||
| + | |When the things are equal to a root of squares of squares: | ||
| + | :<math>\scriptstyle bx=\sqrt{ax^4}</math> | ||
| + | |style="text-align:right;"|כאשר הדברים יהיו שוים אל שרשי צינסו מצינסי | ||
| + | |- | ||
| + | |The number of the things should be multiplied by itself. | ||
| + | |style="text-align:right;"|צריך להכות כמות הדברים בעצמם | ||
| + | |- | ||
| + | |Then, this product should be divided by the number of the squares of squares that are the radicand. | ||
| + | |style="text-align:right;"|ולחלק אותה ההכאה על כמות הצינסי מצינסי הנקובי' היות להם שרש | ||
| + | |- | ||
| + | |The root of the result is equal to the thing. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{x=\sqrt{\frac{b^2}{a}}}}</math> | ||
| + | |style="text-align:right;"|ושרש העולה יבא להיות שוה הדבר | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:a/b=⅗;ab√8=b|618|nOEQ}}Find me two numbers such that the one is a part of the other as 3 is of 5; | ||
| + | :and when the one is multiplied by the other, then this product is multiplied by the the root of 8, it yields the same as the second number. | ||
| + | :I ask: how much will each number be? | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle a:b=3:5\\\scriptstyle\left(a\sdot b\right)\sdot\sqrt{8}=b\end{cases}</math> | ||
| + | |style="text-align:right;"|עשה לי זה החשבון תמצא לי ב' מספרי' שיהיה האחד חלק מהאחר כמו שהוא ג' מה‫'<br> | ||
| + | וכשיוכה האחד באחר ואותה ההכאה תוכה בשרש ח' יעשה כמו המספר השני<br> | ||
| + | אשאל כמה הוא כל אחד מהמספרים{{#annotend:nOEQ}} | ||
| + | |- | ||
| + | | | ||
| + | :This is its rule | ||
| + | |style="text-align:right;"|זהו כללו | ||
| + | |- | ||
| + | | | ||
| + | :Suppose the first number is three things [<math>\scriptstyle{\color{blue}{a=3x}}</math>] and the second is five things [<math>\scriptstyle{\color{blue}{b=5x}}</math>] | ||
| + | |style="text-align:right;"|תניח שהמספר ראשון יהיה ג' דברים והשני יהיה ה' דברים | ||
| + | |- | ||
| + | | | ||
| + | :Now, multiply the one by the other, i.e. 3 things by 5 things; the result is 15 squares. | ||
| + | :<math>\scriptstyle{\color{blue}{3x\sdot5x=15x^2}}</math> | ||
| + | |style="text-align:right;"|עתה תכה האחד באחר דהיינו ג' דברים בה' דברי' עולה ט"ו צינסי | ||
| + | |- | ||
| + | | | ||
| + | :Multiply this product, which is 15 squares, by a root of 8: | ||
| + | |style="text-align:right;"|עתה תכה זאת ההכאה שהיא ט"ו צינסי בשרש ח‫' | ||
| + | |- | ||
| + | | | ||
| + | :Remember that you must convert the 15 squares into a root; you get a root of 225 squares of squares. | ||
| + | |style="text-align:right;"|וזכור כי הנך צריך להשיב הט"ו צינסי אל שרש ויהיה לך שרש מרכ"ה צינסי מצינסי | ||
| + | |- | ||
| + | | | ||
| + | :Multiply it by a root of 8 ; you get a root of 1800 squares of squares. Keep it for one side of the equation. | ||
| + | :<math>\scriptstyle{\color{blue}{15x^2\sdot\sqrt{8}=\sqrt{225x^4\sdot8}=\sqrt{1800x^4}}}</math> | ||
| + | |style="text-align:right;"|ותכם בשרש ח' ויהיה לך שרש מאלף ת"ת צינסי מצינסי ושמרם בעד חלק אחד מההשואה | ||
| + | |- | ||
| + | | | ||
| + | :You have 5 things that are equal to a root of 1800 squares of squares. | ||
| + | :<math>\scriptstyle5x=\sqrt{1800x^4}</math> | ||
| + | |style="text-align:right;"|א"כ יהיה לך ה' דברי' שוים אל שרש אלף ת"ת צינסי מצינסי | ||
| + | |- | ||
| + | | | ||
| + | :Pursue the above said rule: | ||
| + | |style="text-align:right;"|עתה תרדוף כפי הכלל האמור למעלה | ||
| + | |- | ||
| + | | | ||
| + | :Multiply the number of the things, which is 5, by itself; the result is 25. | ||
| + | |style="text-align:right;"|תכה כמות הדברים בעצמם והם ה' ועולה כ"ה | ||
| + | |- | ||
| + | | | ||
| + | :Divide this product, i.e. 25, by the number of the squares of squares that are the radicand, which is 1800; the result is one part of 72. | ||
| + | |style="text-align:right;"|וזאת ההכאה רצוני כ"ה תחלק בכמות הצינסי מצינסי הנקובים להיות להם שרש שהם אלף ת"ת ויבא מזה חלק אחד מע"ב | ||
| + | |- | ||
| + | | | ||
| + | :The root of this part of 72 is the thing. | ||
| + | :<math>\scriptstyle{\color{blue}{x=\sqrt{\frac{5^2}{1800}}=\sqrt{\frac{25}{1800}}=\sqrt{\frac{1}{72}}}}</math> | ||
| + | |style="text-align:right;"|ושרש זה החלק מע"ב יבא להיות הדבר | ||
| + | |- | ||
| + | | | ||
| + | :You assumed the first number is 3 things, so multiply 3 by a root of one part of 72; the result is a root of 9 parts of 72, which is a root of one part of 8, and this is the first number. | ||
| + | :<math>\scriptstyle{\color{blue}{a=3x=3\sdot\sqrt{\frac{1}{72}}=\sqrt{\frac{9}{72}}=\sqrt{\frac{1}{8}}}}</math> | ||
| + | |style="text-align:right;"|ואתה הנחת היות המספר הראשון ג' דברים<br> | ||
| + | א"כ תכה ג' בשרש חלק א' מע"ב ועולה שרש מט' חלקים מע"ב שיבא להיות שרש מא' חלק מח' וככה יבא להיות המספר ראשון | ||
| + | |- | ||
| + | | | ||
| + | :You assumed the second is 5 things, so multiply 5 by a root of one part of 72; the result is a root of 25 parts of 72 and this is the second number. | ||
| + | :<math>\scriptstyle{\color{blue}{b=5x=5\sdot\sqrt{\frac{1}{72}}=\sqrt{\frac{25}{72}}}}</math> | ||
| + | |style="text-align:right;"|והשני הנחת היותו ה' דברי‫'<br> | ||
| + | א"כ תכה ה' בשרש א' חלק מע"ב ועולה שרש מכ"ה חלקים מע"ב וככה יבא להיות המספר השני | ||
| + | |- | ||
| + | |Know that this equation can be returned to the second chapter, and also can be returned to chapter 13: | ||
| + | |style="text-align:right;"|ודע כי זאת ההשואה אפש' להשיבה אל הפרק השני וג"כ אפש' להשיבה אל הפרק הי"ג | ||
| + | |- | ||
| + | |First to chapter 13: by multiplying each side [of the equation] by itself. | ||
| + | |style="text-align:right;"|‫<ref>33r</ref>וראשונה אל הי"ג בהכות כל אחד מהחלקי' בעצמו | ||
| + | |- | ||
| + | |The things become squares. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\left(bx\right)^2=Bx^2}}</math> | ||
| + | |style="text-align:right;"|הדברי' יבאו צינסי | ||
| + | |- | ||
| + | |The root of squares of squares becomes squares of squares. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\left(\sqrt{ax^4}\right)^2=ax^4}}</math> | ||
| + | |style="text-align:right;"|ושרש צינסו מצינסו יבא צינסו מצינסו | ||
| + | |- | ||
| + | |By dividing also each side by a square: | ||
| + | |style="text-align:right;"|ובחלוק ג"כ כל חלק על צינסו | ||
| + | |- | ||
| + | |The squares become numbers. | ||
| + | |style="text-align:right;"|הצינסי {{#annot:term|875,1566|wv2i}}יבאו אל{{#annotend:wv2i}} מספרי‫' | ||
| + | |- | ||
| + | |The squares of squares become squares. | ||
| + | |style="text-align:right;"|והצינסי מצינסי יבאו צינסי | ||
| + | |- | ||
| + | |Thus, it is returned to the second chapter. | ||
| + | |style="text-align:right;"|והיה מושב אל הפרק השני | ||
| + | |- | ||
| + | |If you wish it can be returned to the third chapter: | ||
| + | |style="text-align:right;"|וברצותך להשיבה אל הפרק השלישי | ||
| + | |- | ||
| + | | | ||
| + | :You have 5 things that are equal to squares whose number is a root of 1800; you should divide 5 by a root of 1800 and the result is equal to the thing. | ||
| + | :<math>\scriptstyle{\color{blue}{5x=\sqrt{1800x^4}\;\longrightarrow\;5x=\sqrt{1800}x^2\;\longrightarrow\;x=\frac{5}{\sqrt{1800}}}}</math> | ||
| + | |style="text-align:right;"|יהיו לך ה' דברי' שוים אל כך צינסי כמו שהוא שרש אלף ת"ת ויבא לך לחלק ה' על שרש אלף ת"ת ומה שיעלה שוה הדבר | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== Chapter 29 ==== | ||
| + | |||
| + | |style="width:45%;text-align:right;"|<big>פרק כ"ט</big> | ||
| + | |- | ||
| + | |When the squares are equal to a root of squares: | ||
| + | :<math>\scriptstyle ax^2=\sqrt{bx^2}</math> | ||
| + | |style="text-align:right;"|כאשר הצינסי יהיו שוים אל שרשי צינסי | ||
| + | |- | ||
| + | |The number of the squares should be multiplied by itself. | ||
| + | |style="text-align:right;"|צריך להכות כמות הצינסי בעצמם | ||
| + | |- | ||
| + | |Then, [the number of] the squares that are the radicand should be divided by this product. | ||
| + | |style="text-align:right;"|ולחלוק הצינסי הנקובי' להיות להם שרש על אותה ההכאה | ||
| + | |- | ||
| + | |The root of the result is equal to the thing. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{x=\sqrt{\frac{b}{a^2}}}}</math> | ||
| + | |style="text-align:right;"|ושרשרש העולה יבא לשוות הדבר | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:a/b=⅘;ab=b√8|618|roVS}}Find me two numbers such that the first is a part of the second as 4 is of 5; | ||
| + | :and the one multiplied by the other yields the same as the product of the second by the root of 8. | ||
| + | :I ask: how much will each number be? | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle a:b=4:5\\\scriptstyle a\sdot b=b\sdot\sqrt{8}\end{cases}</math> | ||
| + | |style="text-align:right;"|תמצא לי ב' מספרי' שיהיה הראשון חלק מהשני כמו שד' הוא מה‫'<br> | ||
| + | ומוכה האחד באחר יעשה כמו הכאת השני בשרש ח‫'<br> | ||
| + | אשאל כמה יבא להיות כל אחד מהמספרי‫'{{#annotend:roVS}} | ||
| + | |- | ||
| + | | | ||
| + | :This is its rule: | ||
| + | |style="text-align:right;"|זהו כללו | ||
| + | |- | ||
| + | | | ||
| + | :Suppose the first number is four things [<math>\scriptstyle{\color{blue}{a=4x}}</math>] and the second is five things [<math>\scriptstyle{\color{blue}{b=5x}}</math>]. | ||
| + | |style="text-align:right;"|תניח שהמספר ראשון ד' דברי' והשני יהיה ה' דברי‫' | ||
| + | |- | ||
| + | | | ||
| + | :Now, multiply the first by the second, i.e. 4 things by 5 things; the result is 20 squares. Keep them for one side of the equation. | ||
| + | :<math>\scriptstyle{\color{blue}{4x\sdot5x=20x^2}}</math> | ||
| + | |style="text-align:right;"|עתה תכה הראשו' בשני דהיינו ד' דברי' בה' דברי' ועולה כ' צינסי ושמרם בעד חלק אחד מן חלקי ההשואה | ||
| + | |- | ||
| + | | | ||
| + | :Multiply the second number, which is 5 things, by a root of 8: | ||
| + | |style="text-align:right;"|תכה המספר השני שהוא ה' דברי' בשרש ח‫' | ||
| + | |- | ||
| + | | | ||
| + | :Remember that you must convert the things into roots; you get 25 squares. | ||
| + | |style="text-align:right;"|וזכור כי הנך צריך להשיב הדברי' אל שרשי' ויהיה לך שרש כ"ה צינסי | ||
| + | |- | ||
| + | | | ||
| + | :Multiply them by a root of 8; the result is a root of 200 squares that is equal to 20 squares. | ||
| + | :<math>\scriptstyle{\color{blue}{5x\sdot\sqrt{8}=\sqrt{25x^2\sdot8}=\sqrt{200x^2}=20x^2}}</math> | ||
| + | |style="text-align:right;"|ותכם בשרש ח' ועולה שרש מר' צינסי שהם שוים אל כ' צינסי | ||
| + | |- | ||
| + | | | ||
| + | :Pursue the above said rule: | ||
| + | |style="text-align:right;"|עתה תרדוף כפי הכלל האמור למעלה | ||
| + | |- | ||
| + | | | ||
| + | :Multiply the number of the squares, which is 20, by itself; the result is 400. | ||
| + | |style="text-align:right;"|תכה כמות הצינסי בעצמם והם כ' ועולה ת‫' | ||
| + | |- | ||
| + | | | ||
| + | :Now, divide the number of the squares that are the radicand, which is 200, by the 400; the result is a half. | ||
| + | |style="text-align:right;"|עתה תחלק כמות הצינסי אשר נקבו להיות להם שרשי' שהם ר' על אלו הת' ויבא חצי | ||
| + | |- | ||
| + | | | ||
| + | :The root of a half is the thing. | ||
| + | :<math>\scriptstyle{\color{blue}{x=\sqrt{\frac{200}{20^2}}=\sqrt{\frac{200}{400}}=\sqrt{\frac{1}{2}}}}</math> | ||
| + | |style="text-align:right;"|ושרש החצי יבא להיות הדבר | ||
| + | |- | ||
| + | | | ||
| + | :You assumed the first number is 4 things, so multiply 4 by a root of a half; the result is a root of 8 and this is the first number. | ||
| + | :<math>\scriptstyle{\color{blue}{a=4x=4\sdot\sqrt{\frac{1}{2}}=\sqrt{8}}}</math> | ||
| + | |style="text-align:right;"|ואתה הנחת היות המספר ראשון ד' דברי‫'<br> | ||
| + | א"כ תכה ד' בשרש חצי יעלה שרש ח' וככה יבא לשוות המספר ראשון | ||
| + | |- | ||
| + | | | ||
| + | :You assumed the second is 5 things, so multiply 5 by a root of a half; the result is a root of 12 and a half, and this is the second number. | ||
| + | :<math>\scriptstyle{\color{blue}{b=5x=5\sdot\sqrt{\frac{1}{2}}=\sqrt{12+\frac{1}{2}}}}</math> | ||
| + | |style="text-align:right;"|והשני הנחת ה' דברים<br> | ||
| + | א"כ תכה ה' בשרש חצי ועולה שרש מי"ב וחצי וככה יבא להיות המספר השני | ||
| + | |- | ||
| + | |Know that this equation can be returned to chapter 13, by multiplying each side [of the equation] by itself, then dividing it by roots; it is converted to squares of squares equal squares. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{Ax^4=bx^2}}</math> | ||
| + | |style="text-align:right;"|<big>ודע</big> כי זאת ההשואה אפשר להשיבה אל הפרק הי"ג בהכות כל חלק בעצמו ולחלקו בשרשי' ויבאו צינסי מצינסי שוים אל צינסי | ||
| + | |- | ||
| + | |Or, if you wish, divide again by things; it is returned to the eighth chapter. | ||
| + | |style="text-align:right;"|ואם רצית לחלק אח"כ על דברי' תשוב אל הפרק השמיני | ||
| + | |- | ||
| + | |Or, if you wish, divide it by squares; it is returned to the second chapter, i.e. numbers equal squares. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{Ax^2=b}}</math>. | ||
| + | |style="text-align:right;"|וברצותך לחלקה על צינסי תשוב אל הפרק השני דהיינו מספרי' שוים אל צינסי | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== Chapter 30 ==== | ||
| + | |||
| + | |style="width:45%;text-align:right;"|<big>פרק שלשים</big> | ||
| + | |- | ||
| + | |When the squares are equal to a root of cubes: | ||
| + | :<math>\scriptstyle bx^2=\sqrt{ax^3}</math> | ||
| + | |style="text-align:right;"|כאשר הצינסי יהיו שוים אל שרשי המעוקבי‫' | ||
| + | |- | ||
| + | |The number of the squares should be multiplied by itself. | ||
| + | |style="text-align:right;"|צריך להכות כמות הצינסי בעצמם | ||
| + | |- | ||
| + | |Then, the number of the cubes that are the radicand should be divided by this product; the result is equal to the thing. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{x=\frac{a}{b^2}}}</math> | ||
| + | |style="text-align:right;"|ולחלק כמות המעוקבי' הנקובי' להיות להם שרש על אותה ההכאה והעולה מזה ככה ישוה הדבר | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:a/b=⅔;a√a=b²|618|LtCT}}Find me two numbers such that one is a part of the other as 2 is of 3; | ||
| + | :and when the first is multiplied by its root it yields the same as the product of the second by itself. | ||
| + | :I ask: how much will each number be? | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle a:b=2:3\\\scriptstyle a\sdot\sqrt{a}=b^2\end{cases}</math> | ||
| + | |style="text-align:right;"|תמצא לי ב' מספרי' שיהיה האחד חלק מהאחר כמו ב' מג‫'<br> | ||
| + | ובהכות הראשו' בשרשו יעשה כמו הכאת השני בעצמו<br> | ||
| + | אשאל כמה יבא להיות כל אחד מן המספרים{{#annotend:LtCT}} | ||
| + | |- | ||
| + | | | ||
| + | :This is its rule: | ||
| + | |style="text-align:right;"|וזהו כללו | ||
| + | |- | ||
| + | | | ||
| + | :Suppose the first number is two things [<math>\scriptstyle{\color{blue}{a=2x}}</math>] and the other is three things [<math>\scriptstyle{\color{blue}{b=3x}}</math>]. | ||
| + | |style="text-align:right;"|תניח שהמספר ראשון היה ב' דברי' והאחר ג' דברי‫' | ||
| + | |- | ||
| + | | | ||
| + | :Multiply the first, which is 2 things, by its root: | ||
| + | |style="text-align:right;"|תכה הראשון שהוא ב' דברי' בשרשם <s>שהוא</s> | ||
| + | |- | ||
| + | | | ||
| + | :Remember that you must convert the 2 things into roots; you get a root of 4 squares. | ||
| + | |style="text-align:right;"|זכור לך כי אתה צריך להשיב ב' דברי' אל שרשי' ויהיה לך שרש מד' צינסי | ||
| + | |- | ||
| + | | | ||
| + | :You should multiply it by a root of 2 things; the result is a root of 8 cubes. Keep it for one side of the equation. | ||
| + | :<math>\scriptstyle{\color{blue}{2x\sdot\sqrt{2x}=\sqrt{4x^2\sdot2x}=\sqrt{8x^3}}}</math> | ||
| + | |style="text-align:right;"|אשר אתה צריך להכות ‫<ref>33v</ref>בשרש ב' דברי' ועולה שרש ח' מעוקב ושמר זה בעד חלק אחד מההשואה | ||
| + | |- | ||
| + | | | ||
| + | :Now, multiply the other number, which is 3 things, by itself; the result is 9 squares that are equal to a root of [8 cubes]. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(3x\right)^2=9x^2=\sqrt{8x^3}}}</math> | ||
| + | |style="text-align:right;"|עתה תכה המספר האחר שהוא ג' דברי' בעצמו ועולה ט' צינסי שהם שוים אל שרש מעוקב ח‫' | ||
| + | |- | ||
| + | | | ||
| + | :Pursue the above given rule: | ||
| + | |style="text-align:right;"|עתה תרדוף כפי הכלל הנתון למעלה | ||
| + | |- | ||
| + | | | ||
| + | :Multiply the number of the squares, which is 9, by itself; the result is 81. | ||
| + | |style="text-align:right;"|תכה כמות הצינסי בעצמם שהם ט' ועולה פ"א | ||
| + | |- | ||
| + | | | ||
| + | :Now, divide the number of the cubes that are the radicand, which is 8, by 81; the result is 8 parts of 81 and this is the thing. | ||
| + | :<math>\scriptstyle{\color{blue}{x=\frac{8}{9^2}=\frac{8}{81}}}</math> | ||
| + | |style="text-align:right;"|עתה תחלק כמות המעוקבי' הנקובי' להיות להם שרש שהם ח' על אלו הפ"א ויבא מזה ח' חלקי' מפ"א וככה שוה הדבר | ||
| + | |- | ||
| + | | | ||
| + | :You assumed the first number is 2 things, so multiply 2 by 8 parts of 81; the result is 16 parts of 81 and this is the first number. | ||
| + | :<math>\scriptstyle{\color{blue}{a=2x=2\sdot\frac{8}{81}=\frac{16}{81}}}</math> | ||
| + | |style="text-align:right;"|ואתה הנחת היות המספר ראשון ב' דברי‫'<br> | ||
| + | א"כ תכה ב' בח' חלקי' מפ"א ועולה י"ו חלקי' מפ"א וככה הוא המספר ראשון | ||
| + | |- | ||
| + | | | ||
| + | :You assumed the second is 3 things, so multiply 3 by 8 parts of 81; the result is 24 parts of 81 and this is the second number. | ||
| + | :<math>\scriptstyle{\color{blue}{b=3x=3\sdot\frac{8}{81}=\frac{24}{81}}}</math> | ||
| + | |style="text-align:right;"|והשני הנחת היותו ג' דברי‫'<br> | ||
| + | א"כ תכה ג' בח' חלקי' מפ"א ועולה כ"ד חלקי' מפ"א וככה הוא המספר השני | ||
| + | |- | ||
| + | |Know that this equation can be returned to the tenth chapter, by dividing by roots: | ||
| + | |style="text-align:right;"|<big>ודע</big> כי זאת ההשואה אפשר להשיבו אל הפרק העשירי בחלוק על שרשים | ||
| + | |- | ||
| + | | | ||
| + | :Since the 9 squares are a root of 81 squares that are equal to [a root of 8] cubes. | ||
| + | :<math>\scriptstyle{\color{blue}{9x^2=\sqrt{81}x^2=\sqrt{8x^3}}}</math> | ||
| + | |style="text-align:right;"|מפני כי ט' צינסי הם שרש מפ"א צינסי אשר הם שוים אל ח' שרשי' מעו‫' | ||
| + | |- | ||
| + | | | ||
| + | :Hence, by multiplying 9 squares that are a root of 81 squares by themselves, the result is 81 squares of squares. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(9x^2\right)=\left(\sqrt{81}x^2\right)=81x^4}}</math> | ||
| + | |style="text-align:right;"|א"כ בהכאת ט' צינסי בעצמם אשר הם שרש מפ"א צינסי <sup>מצינסי</sup> עושים פ"א צינסי מצינסי | ||
| + | |- | ||
| + | | | ||
| + | :By multiplying a root of 8 cubes by itself, the result is 8 cubes. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(\sqrt{8x^3}\right)=8x^3}}</math> | ||
| + | |style="text-align:right;"|ולהכות שרש ח' מעוקבי' בעצמו עושה ח' מעוקבי‫' | ||
| + | |- | ||
| + | | | ||
| + | :Therefore, you get 81 squares of squares equal 8 cubes; and this is the method of dividing by roots. | ||
| + | :<math>\scriptstyle{\color{blue}{9x^2=\sqrt{8x^3}\;\longrightarrow\;81x^4=8x^3}}</math> | ||
| + | |style="text-align:right;"|א"כ יהיה לך פ"א צינסי מצינסי שוים אל ח' מעוקבי' וזהו האופן לחלק בשרשי‫' | ||
| + | |- | ||
| + | |When we wish to divide the equation by a thing, it brings you to the ninth chapter. | ||
| + | |style="text-align:right;"|וברצותנו לחלק זאת ההשואה על דברי' תביאך אל הפרק התשיעי | ||
| + | |- | ||
| + | |By dividing it by a square, it brings you to the third chapter. | ||
| + | |style="text-align:right;"|ובחלוק אותה על צינסי תביאך אל הפרק השלישי | ||
| + | |- | ||
| + | |If you divide it by a cube, it brings you to chapter 1. | ||
| + | |style="text-align:right;"|ואם תחלקנה על מעו' תביאך אל פרק א‫' | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== Chapter 31 ==== | ||
| + | |||
| + | |style="text-align:right;"|<big>פרק ל"א</big> | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|עוד רצוני להראותך באופן אחר | ||
| + | |- | ||
| + | |When the cubes are equal to a root of squares: | ||
| + | :<math>\scriptstyle ax^3=\sqrt{bx^2}</math> | ||
| + | |style="text-align:right;"|כאשר המעוקבי' יהיו שוים אל שרשי צינסי | ||
| + | |- | ||
| + | |The number of the cubes should be multiplied by itself. | ||
| + | |style="text-align:right;"|צריך להכות כמות המעוקבי' בעצמם | ||
| + | |- | ||
| + | |Then, the number of the squares that are the radicand should be divided by this product. | ||
| + | |style="text-align:right;"|ולחלק כמות הצינסי הנקובי' להיות להם שרש על אותה ההכאה | ||
| + | |- | ||
| + | |Extract the root of the root of the quotient and this is the thing. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{x=\sqrt[4]{\frac{b}{a^2}}}}</math> | ||
| + | |style="text-align:right;"|ו{{#annot:term|795|Cvo2}}תקח שרש השרש מ{{#annotend:Cvo2}}העולה בחלוק וככה ישוה הדבר | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:(a·⅔a)·a=a√8|618|iQR6}}Find me a number, such that when it is multiplied by its two thirds, and this product is multiplied by that number, it yields the same as the product of that number by the root of 8. | ||
| + | :I ask: how much will the number be? | ||
| + | :<math>\scriptstyle\left(a\sdot\frac{2}{3}a\right)\sdot a=a\sdot\sqrt{8}</math> | ||
| + | |style="text-align:right;"|תמצא לי מספר אחד שכשיוכה בשני שלישיו ואותה ההכאה תוכה במספר האמור יעשה כמו הכאת המספר ההוא בשרש ח‫'<br> | ||
| + | אשאל כמה הוא כל אחד מהמספרי‫'{{#annotend:iQR6}} | ||
| + | |- | ||
| + | | | ||
| + | :This is its rule: | ||
| + | |style="text-align:right;"|זהו כללו | ||
| + | |- | ||
| + | | | ||
| + | :Suppose the number is one thing [<math>\scriptstyle{\color{blue}{a=x}}</math>]. | ||
| + | |style="text-align:right;"|תניח שהמספר הוא דבר אחד | ||
| + | |- | ||
| + | | | ||
| + | :Multiply one thing by its 2-thirds; the result is 2-thirds of a square. | ||
| + | |style="text-align:right;"|תכה עתה דבר אחד בב' שלישיו ועולה ב' שלישי צינסו | ||
| + | |- | ||
| + | | | ||
| + | :Multiply this product by the said number, i.e. by one thing; the result is 2-thirds of a cube. Keep it for one side of the equation. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(x\sdot\frac{2}{3}x\right)\sdot x=\frac{2}{3}x^2\sdot x=\frac{2}{3}x^3}}</math> | ||
| + | |style="text-align:right;"|וזאת ההכאה תכה במספר האמור דהיינו בדבר אחד ועולה ב' שלישי מעוקב ושמור זה בעד חלק אחד מההשואה | ||
| + | |- | ||
| + | | | ||
| + | :Then, multiply the said number, i.e. one thing, by a root of 8: | ||
| + | |style="text-align:right;"|אח"כ תכה המספר האמור רצוני דבר אחד בשרש ח‫' | ||
| + | |- | ||
| + | | | ||
| + | :Remember to convert the things into a root; you get a root of 1 square. | ||
| + | |style="text-align:right;"|ותזכור להשיב הדברי' אל שרש ויהיה לך שרש מא' צינסו | ||
| + | |- | ||
| + | | | ||
| + | :Multiply it by a root of 8; the result is a root of 8 squares, which is equal to 2-thirds of a cube. | ||
| + | :<math>\scriptstyle{\color{blue}{x\sdot\sqrt{8}=\sqrt{8x^2}=\frac{2}{3}x^3}}</math> | ||
| + | |style="text-align:right;"|אשר תכהו בשרש ח' ועולה שרש ח' צינסי שהם שוים אל ב' שלישי מעוקב | ||
| + | |- | ||
| + | | | ||
| + | :Pursue the above mentioned rule: | ||
| + | |style="text-align:right;"|עתה תרדוף כפי הכלל האמור למעלה | ||
| + | |- | ||
| + | | | ||
| + | :Multiply the number of the cubes, which is 2-thirds, by itself; the result is 4-ninths. | ||
| + | |style="text-align:right;"|תכה כמות המעוקבי' בעצמו והוא ב' שלישי' ועולה ד' תשיעיות | ||
| + | |- | ||
| + | | | ||
| + | :Divide the number of the squares that are the radicand, which is 8, by the 4-ninths; the result is 18. | ||
| + | |style="text-align:right;"|ותחלק כמות הצינסי הנקובי' להיות להם שרש והם ח' על אלו הד' תשיעיו' ויבא מזה י"ח | ||
| + | |- | ||
| + | | | ||
| + | :A root of a root of 18 is the thing and so is the said number. | ||
| + | :<math>\scriptstyle{\color{blue}{x=\sqrt[4]{\frac{8}{\frac{2}{3}^2}}=\sqrt[4]{\frac{8}{\frac{4}{9}}}=\sqrt[4]{18}}}</math> | ||
| + | |style="text-align:right;"|ושרש השרש מי"ח יבא להיות הדבר וכן הוא המספר האמור | ||
| + | |- | ||
| + | |Know that this equation can be returned to chapter 11, by dividing by roots: | ||
| + | |style="text-align:right;"|<big>ודע</big> כי זאת ההשואה אפשר להשיבה אל הפרק הי"א בזה האופן בחלק בשרשי‫' | ||
| + | |- | ||
| + | |As you mentioned roots in one side, convert the other side into roots: so, multiply the cubes by themselves; you get a root of squares that is equal to a root of squares of squares [of squares]. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{ax^3=\sqrt{bx^2}\;\longrightarrow\;\sqrt{\left(ax^3\right)^2}=\sqrt{bx^2}\;\longrightarrow\;\sqrt{Ax^6}=\sqrt{bx^2}}}</math> | ||
| + | |style="text-align:right;"|כי כמו שנקבת שרשי' <sup>בחלק</sup> בצד האחד כן ג"כ תשיב <sup>החלק</sup> הצד האחר אל שרשים תכה א"כ המעוקבי' בעצמם ויהיה לך שרשי צינסי שוים אל שרשי צינסי מצינסי | ||
| + | |- | ||
| + | |Since cubes by cubes generate cubes of cubes that are squares of squares of squares. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\left(ax^3\right)^2=ax^3\sdot ax^3=ax^2\sdot ax^2\sdot ax^2}}</math> | ||
| + | |style="text-align:right;"|‫<ref>34r</ref>מפני כי מעו' במעו' עושה {{#annot:term|689|Ae4R}}מעו' המעו'{{#annotend:Ae4R}} אשר הם באי' להיות {{#annot:term|689|R4d1}}צינסי דצינסי דצינסי{{#annotend:R4d1}} | ||
| + | |- | ||
| + | |So, by dividing by a root the result is squares equal squares of squares of squares. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\sqrt{Ax^6}=\sqrt{bx^2}\;\longrightarrow\;Ax^6=bx^2}}</math> | ||
| + | |style="text-align:right;"|א"כ {{#annot:term|157,1223|K0ln}}בחלוק ב{{#annotend:K0ln}}שרשי' יבא צינסו שוה אל {{#annot:term|689|ioCC}}צינסו דצינסו מצינסו{{#annotend:ioCC}} | ||
| + | |- | ||
| + | |By dividing by a square the result is a number that is equal to squares of squares. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\sqrt{Ax^6}=\sqrt{bx^2}\;/\div x^2\;\longrightarrow\;Ax^4=b}}</math> | ||
| + | |style="text-align:right;"|אשר זה בחלוק בצינסי יבא ממנו מספר שוה לצינסו מצינסו | ||
| + | |- | ||
| + | |In this way that you have seen it can be returned properly. | ||
| + | |style="text-align:right;"|ובאופן הזה אשר ראית אפשר להשיבה מאד היטב | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== Chapter 32 ==== | ||
| + | |||
| + | |style="text-align:right;"|<big>פרק ל"ב</big> | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|עשה לי זה החשבון אשר אומר אליך פה בקרוב | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|אבל קודם זה רצוני להבינך טבע זה הפרק וזה הוא | ||
| + | |- | ||
| + | |When squares of squares are equal to a root of squares: | ||
| + | :<math>\scriptstyle ax^4=\sqrt{bx^2}</math> | ||
| + | |style="text-align:right;"|כאשר צינסי מצינסי יהיו שוים אל שרשי' מצינסי | ||
| + | |- | ||
| + | |The number of the squares of squares should be multiplied by itself. | ||
| + | |style="text-align:right;"|צריך להכות כמות הצינסי מצינסי בעצמם | ||
| + | |- | ||
| + | |Then, the number of the squares that are the radicand should be divided by this product. | ||
| + | |style="text-align:right;"|ולחלק כמות הצינסי הנקובי' להיות להם שרש על אותה הכאה | ||
| + | |- | ||
| + | |The square root of the cube root of the result is equal to the thing. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{x=\sqrt[6]{\frac{b}{a^2}}}}</math> | ||
| + | |style="text-align:right;"|ושרש המרובע משרש המעו' ממה שיעלה יבא לשוות הדבר | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:a/b=⅔;(ab)²=b√8|618|6dSA}}Find me two numbers such that the first is a part of the second as 2 is of 3; | ||
| + | :and when the smaller is multiplied by the greater, and this product is multiplied by itself, it yields the same as the product of the second by the root of 8. | ||
| + | :I ask: how much will each number be? | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle a:b=2:3\\\scriptstyle\left(a\sdot b\right)^2=b\sdot\sqrt{8}\end{cases}</math> | ||
| + | |style="text-align:right;"|תמצא לי שני מספרים שיהיה הראשון חלק מהשני כמו שהוא ב' מג‫'<br> | ||
| + | ויוכה הקטון בגדול ואותה ההכאה תוכה בעצמה יעשה כמו הכאת השני בשרש ח‫'<br> | ||
| + | אשאל כמה יבא להיות כל אחד מהמספרי‫'{{#annotend:6dSA}} | ||
| + | |- | ||
| + | | | ||
| + | :This is its rule: | ||
| + | |style="text-align:right;"|זהו כללו | ||
| + | |- | ||
| + | | | ||
| + | :Suppose the first number is two things [<math>\scriptstyle{\color{blue}{a=2x}}</math>] and the second is three things [<math>\scriptstyle{\color{blue}{b=3x}}</math>]. | ||
| + | |style="text-align:right;"|תשים שהמספר ראשון יהיה <sup>ב'</sup> דברי' <s>אחר</s> והשני ג' דברי‫' | ||
| + | |- | ||
| + | | | ||
| + | :Multiply the smaller by the greater, which is 2 things by 3 things; the result is 6 squares. | ||
| + | |style="text-align:right;"|תכה הקטון בגדול וזהו ב' דברי' בג' דברי' ועולה ו' צינסי | ||
| + | |- | ||
| + | | | ||
| + | :Then, multiply this product, which is 6 squares, [by itself]; the result is 36 squares of squares. Keep it for one [side] of the equation. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(2x\sdot3x\right)^2=\left(6x^2\right)^2=36x^4}}</math> | ||
| + | |style="text-align:right;"|אח"כ תכה זאת ההכאה שהיא ו' צינסי ועולה ל"ו צינסי מצינסי ושמור זה בעד אחד מההשואה | ||
| + | |- | ||
| + | | | ||
| + | :Multiply the second that is the greater, which is 3 things, by a root of 8. | ||
| + | |style="text-align:right;"|אח"כ תכה השני שהוא הגדול שהוא ג' דברי' בשרש ח‫' | ||
| + | |- | ||
| + | | | ||
| + | :Remember that you must convert 3 things into a root; you get a root of 9 squares. | ||
| + | |style="text-align:right;"|וזכור כי אתה צריך להשיב ג' דברי' אל שרש ויהיה לך שרש מט' צינסי | ||
| + | |- | ||
| + | | | ||
| + | :Multiply it by a root of 8; the result is a root of 72 squares that is equal to [36] squares of squares. | ||
| + | :<math>\scriptstyle{\color{blue}{3x\sdot\sqrt{8}=\sqrt{9x^2\sdot8}=\sqrt{72x^2}=3{\color{red}{6}}x^4}}</math> | ||
| + | |style="text-align:right;"|ותכהו על שרש ח' ועולה שרש מע"ב צינסי שהם שוים אל ג' צינסי מצינסי | ||
| + | |- | ||
| + | | | ||
| + | :Pursue the above mentioned rule: | ||
| + | |style="text-align:right;"|עתה תרדוף כפי הכלל האמור למעלה | ||
| + | |- | ||
| + | | | ||
| + | :Multiply the number of the squares of squares, which is 36, by itself; the result is 1296. | ||
| + | |style="text-align:right;"|תכה כמות הצינסי מצינסי בעצמם שזהו ל"ו בעצמם ועולה אלף ורצ"ו | ||
| + | |- | ||
| + | | | ||
| + | :Divide the number of the squares that are the radicand, which is 72, by 1296; the result is one part of 18. | ||
| + | |style="text-align:right;"|וחלק כמות הצינסי הנקובי' להיות להם שרש שהם ע"ב על אלו אלף ורצ"ו שיבא ממנו חלק אחד מי"ח | ||
| + | |- | ||
| + | | | ||
| + | :The square root of the cube root, or say: the cube root of the square root of one part of 18 is equal to the thing. | ||
| + | :<math>\scriptstyle{\color{blue}{x=\sqrt[6]{\frac{72}{36^2}}=\sqrt[6]{\frac{72}{1296}}=\sqrt[6]{\frac{1}{18}}}}</math> | ||
| + | |style="text-align:right;"|ושרש המרובע משרש המעו' או אמור השרש המעו' משרש המרובע מחלק אחד מי"ח יבא לשוות הדבר | ||
| + | |- | ||
| + | | | ||
| + | :You assumed the first number is 2 things, so multiply 2 by a square root of a cube root of one part of 18; the result is a cube root of a square root of 64 parts of 18, which is a square root of a cube root of 3 and 5-ninths and this is the first number. | ||
| + | :<math>\scriptstyle{\color{blue}{a=2x=2\sdot\sqrt[6]{\frac{1}{18}}=\sqrt[6]{\frac{64}{18}}=\sqrt[6]{3+\frac{5}{9}}}}</math> | ||
| + | |style="text-align:right;"|ואתה הנחת היות המספר הראשון ב' דברי‫'<br> | ||
| + | א"כ תכה ב' בשרש מרובע משרש מעו' מחלק א' מי"ח ועולה שרש מעו' משרש מרובע מס"ד חלקי' מי"ח אשר הם שרש מרובע משרש מעו' מג' וה' תשיעיות וככה יבא להיות המספר ראשון | ||
| + | |- | ||
| + | | | ||
| + | :You assumed the second is 3 things, so multiply 3 by a cube root of a square root of one part of 18; the result is a square root of a cube root of 729 parts of 18, which is [a square root of a cube root of] 40 and a half and this is the second number. | ||
| + | :<math>\scriptstyle{\color{blue}{b=3x=3\sdot\sqrt[6]{\frac{1}{18}}=\sqrt[6]{\frac{729}{18}}=\sqrt[6]{40+\frac{1}{2}}}}</math> | ||
| + | |style="text-align:right;"|והשני הנחת היותו ג' דברי‫'<br> | ||
| + | א"כ תכה ג' בשרש מעו' משרש מרובע מחלק א' מי"ח ועולה שרש מרובע משרש מעו' מתשכ"ט חלקי' מי"ח שזהו מ' וחצי וככה הוא המספר השני | ||
| + | |- | ||
| + | |Know that this equation can be returned to chapter 21. | ||
| + | |style="text-align:right;"|ודע כי זאת ההשואה אפשר להשיבה אל הפרק הכ"א | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== Chapter 33 ==== | ||
| + | |||
| + | |style="text-align:right;"|<big>פרק ל"ג</big> | ||
| + | |- | ||
| + | |When the cubes are equal to a root of cubes: | ||
| + | :<math>\scriptstyle ax^3=\sqrt{bx^3}</math> | ||
| + | |style="text-align:right;"|כאשר המעוקבי' יהיו שוים אל שרשי מעוקבי‫' | ||
| + | |- | ||
| + | |The number of the cubes should be multiplied by itself. | ||
| + | |style="text-align:right;"|צריך להכות כמות המעוקבי' בעצמם | ||
| + | |- | ||
| + | |Then, the number of the cubes that are the radicand should be divided by this product. | ||
| + | |style="text-align:right;"|ולחלק כמות המעוק' הנקובי' להיות להם שרש באותה ההכאה | ||
| + | |- | ||
| + | |The cube root of the result is equal to the thing. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{x=\sqrt[3]{\frac{b}{a^2}}}}</math> | ||
| + | |style="text-align:right;"|ושרש המעו' מהעולה מזה יבא לשוות הדבר | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:a/b=⅔;a²b=b√b|618|DcQa}}Find me two numbers such that the one is a part of the other as 2 is of 3; | ||
| + | :and when the first is multiplied by itself and this product is multiplied by the second, it yields the same as the product of the second by its root. | ||
| + | :I ask: how much will each number be? | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle a:b=2:3\\\scriptstyle a^2\sdot b=b\sdot\sqrt{b}\end{cases}</math> | ||
| + | |style="text-align:right;"|תמצא לי שני מספרי' שיהיה האחד חלק מהשני כמו שב' הוא מג‫'<br> | ||
| + | ומוכה הראשון בעצמו ואותה ההכאה תוכה במספר השני יעשה ‫<ref>34v</ref>כמו הכאת השני בשרשו<br> | ||
| + | אשאל כמה יבא להיות כל אחד מהמספרי‫'{{#annotend:DcQa}} | ||
| + | |- | ||
| + | | | ||
| + | :This is its rule: | ||
| + | |style="text-align:right;"|זהו כללו | ||
| + | |- | ||
| + | | | ||
| + | :Suppose the first number is two things [<math>\scriptstyle{\color{blue}{a=2x}}</math>] and the other is three things [<math>\scriptstyle{\color{blue}{b=3x}}</math>]. | ||
| + | |style="text-align:right;"|תניח שהמספר ראשון יהיה ב' דברי' והאחר יבא להיות ג' דברים | ||
| + | |- | ||
| + | | | ||
| + | :Now, multiply the first, which is 2 things, by itself; the result is 4 squares. | ||
| + | |style="text-align:right;"|עתה תכה הראשון שהוא ב' דברים בעצמם ועולה ד' צינסי | ||
| + | |- | ||
| + | | | ||
| + | :Multiply this product, which is 4 squares, by the second number, which is 3 things; the result is 12 cubes. Keep them for one side of the equation. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(2x\right)^2\sdot3x=4x^2\sdot3x=12x^3}}</math> | ||
| + | |style="text-align:right;"|עתה תכה זאת ההכאה שהיא ד' צינסי במספר השני שהוא ג' דברי' ועולה י"ב מעוקבי' ושמרם בעד חלק אחד מההשואה | ||
| + | |- | ||
| + | | | ||
| + | :Then, multiply the second number, which is 3 things by a root of 3 things: | ||
| + | |style="text-align:right;"|אח"כ תכה המספר השני שהוא ג' דברים בשרש ג' דברי‫' | ||
| + | |- | ||
| + | | | ||
| + | :Remember that you must convert the 3 things into a root; you get a root of 9 squares. | ||
| + | |style="text-align:right;"|ותזכור כי הנך צריך להשיב ג' דברי' אל שרש ויהיה לך שרש מט' צינסי | ||
| + | |- | ||
| + | | | ||
| + | :Multiply this root of 9 squares by a root of 3 things; the result is a root of [27] cubes that is equal to 12 cubes. | ||
| + | :<math>\scriptstyle{\color{blue}{3x\sdot\sqrt{3x}=\sqrt{9x^2\sdot3x}=\sqrt{{\color{red}{27}}x^3}=12x^3}}</math> | ||
| + | |style="text-align:right;"|וזה השרש מט' צינסי תכה בשרש ג' דברי' ועולה שרש מרע"ה מעוקבי' שהם שוים אל י"ב מעוקבי‫' | ||
| + | |- | ||
| + | | | ||
| + | :Pursue the above mentioned rule: | ||
| + | |style="text-align:right;"|עתה תרדוף כפי הכלל האמור למעלה | ||
| + | |- | ||
| + | | | ||
| + | :Multiply the number of the cubes, which is 12, by itself; the result is 144. | ||
| + | |style="text-align:right;"|תכה כמות המעו' בעצמם וזהו י"ב עולה קמ"ד | ||
| + | |- | ||
| + | | | ||
| + | :Divide the number of the cubes that are the radicand, which is [27], by 144; the result is 3 parts of 16. | ||
| + | |style="text-align:right;"|ותחלק כמות המעוקבי' הנקובי' להיות להם שרש וזהו י"ב על קמ"ד ויבא מזה ג' חלקי' מי"ו | ||
| + | |- | ||
| + | | | ||
| + | :The cube root of 3 parts of 16 is equal to the thing. | ||
| + | :<math>\scriptstyle{\color{blue}{x=\sqrt[3]{\frac{{\color{red}{27}}}{12^2}}=\sqrt[3]{\frac{{\color{red}{27}}}{144}}=\sqrt[3]{\frac{3}{16}}}}</math> | ||
| + | |style="text-align:right;"|ושרש מעו' מג' חלקי' מי"ו יבא לשוות הדבר | ||
| + | |- | ||
| + | | | ||
| + | :You assumed the first number is 2 things, so multiply 2 by a cube root of 3 parts of 16; the result is a cube root of one and a half and this is the first number. | ||
| + | :<math>\scriptstyle{\color{blue}{a=2x=2\sdot\sqrt[3]{\frac{3}{16}}=\sqrt[3]{1+\frac{1}{2}}}}</math> | ||
| + | |style="text-align:right;"|ואתה הנחת היות המספר ראשון ב' דברי‫'<br> | ||
| + | א"כ תכה ב' בשרש מעו' מג' חלקי' מי"ו ועולה שרש מעו' <s>מחצי</s> מאחד וחצי וככה יבא להיות המספר ראשון | ||
| + | |- | ||
| + | | | ||
| + | :You assumed the second is 3 things, so multiply 3 by a cube root of 3 parts of 16; the result is a cube root of [five] and 5 parts of 16 and this is the second number. | ||
| + | :<math>\scriptstyle{\color{blue}{b=3x=3\sdot\sqrt[3]{\frac{3}{16}}=\sqrt[3]{{\color{red}{5+\frac{1}{16}}}}}}</math> | ||
| + | |style="text-align:right;"|והשני הנחת היותו ג' דברי‫'<br> | ||
| + | א"כ תכה ג' בשרש מעו' מג' חלקי' מי"ו ועולה שרש מעו' מה' חלקי' מי"ו וככה הוא המספר השני | ||
| + | |- | ||
| + | |Know that this equation can be returned to the seventh chapter, by multiplying each side [of the equation] by itself. | ||
| + | |style="text-align:right;"|<big>ודע</big> כי זאת ההשואה אפשר להשיבה אל הפרק השביעי בהכות כל אחד מהחלקי' בעצמו | ||
| + | |- | ||
| + | |By dividing the product by a cube, the result is numbers equal cubes. | ||
| + | |style="text-align:right;"|ו{{#annot:term|783,1566|o62i}}יבא בחלוק{{#annotend:o62i}} ההכאה על מעוקבי' מספרי' שוים אל מעוקבי‫' | ||
| + | |- | ||
| + | |A root of cubes by a root of cubes generate cubes. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\left(\sqrt{bx^3}\right)^2=bx^3}}</math> | ||
| + | |style="text-align:right;"|ועל שרשי מעוקבי' בשרשי מעו' עושה מעוקבי‫' | ||
| + | |- | ||
| + | |Cubes by themselves generate cubes of cubes. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\left(ax^3\right)^2=Ax^6}}</math> | ||
| + | |style="text-align:right;"|ומעוקבי' בעצמם עושה {{#annot:term|689|nLSh}}מעו' ממעו‫'{{#annotend:nLSh}} | ||
| + | |- | ||
| + | |Hence, by dividing cubes by cubes the result is numbers. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{bx^3\div x^3=b}}</math> | ||
| + | |style="text-align:right;"|א"כ בחלוק מעו' על מעו' יעלה מהם מספרים | ||
| + | |- | ||
| + | |And cubes of cubes by cubes the result is cubes. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{Ax^6\div x^3=Ax^3}}</math> | ||
| + | |style="text-align:right;"|ו{{#annot:term|689|3zl2}}מעוקבי' ממעוקבי'{{#annotend:3zl2}} על מעוקבי' יבא מהם מעוקבי‫' | ||
| + | |- | ||
| + | |By dividing by squares, the result is things equal squares of squares, so it is returned to chapter 12. | ||
| + | |style="text-align:right;"|ובחלוק על צינסי יבא מזה דבר שוה אל צינסו מצינסו ויבא להיותה מושבת אל הפרק הי"ב | ||
| + | |- | ||
| + | |We proceed according to the aforesaid chapter. | ||
| + | |style="text-align:right;"|ונעשה עם הפרק האמור למעלה | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== Chapter 34 ==== | ||
| + | |||
| + | |style="text-align:right;"|<big>פרק ל"ד</big> | ||
| + | |- | ||
| + | |When the cubes are equal to a root of squares of squares: | ||
| + | :<math>\scriptstyle bx^3=\sqrt{ax^4}</math> | ||
| + | |style="text-align:right;"|כאשר המעו' הם שוים לשרשי צינסי מצינסי | ||
| + | |- | ||
| + | |The number of the cubes should be multiplied by itself. | ||
| + | |style="text-align:right;"|צריך להכות כמות המעוקבי' בעצמם | ||
| + | |- | ||
| + | |Then, the number of squares of squares that are the radicand should be multiplied by this product. | ||
| + | |style="text-align:right;"|ולחלק כמות הצינסי מצינסי הנקובי' היות להם שרש על אותה ההכאה | ||
| + | |- | ||
| + | |The root of the result is equal to the thing. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{x=\sqrt{\frac{a}{b^2}}}}</math> | ||
| + | |style="text-align:right;"|והעולה מזה הנה שרשו ישוה הדבר | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:a/b=⅗;a²b=b²√8|618|YrhT}}Find me two numbers such that the one is a part of the other as 3 is of 5; | ||
| + | :and the the first is multiplied by the other, then this product is multiplied by the second number, it yields the same as the product of the second by itself, then the product is multiplied by the root of 8. | ||
| + | :I ask: how much will each number be? | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle a:b=3:5\\\scriptstyle a^2\sdot b=b^2\sdot\sqrt{8}\end{cases}</math> | ||
| + | |style="text-align:right;"|עשה לי זה החשבון תמצא לי שני מספרי' שיהיה האחד חלק מהאחר כמו שג' הם מה‫'<br> | ||
| + | ומוכה הראשון בעצמו ואותה ההכאה תוכה <s>בשני</s> במספר השני יעשה כמו הכאת השני בעצמו ואותה ההכאה תוכה בשרש ח‫'<br> | ||
| + | אשאל כמה יבא להיות כל אחד מהמספרי‫'{{#annotend:YrhT}} | ||
| + | |- | ||
| + | | | ||
| + | :This is its rule: | ||
| + | |style="text-align:right;"|זהו כללו | ||
| + | |- | ||
| + | | | ||
| + | :Suppose the first number is three things [<math>\scriptstyle{\color{blue}{a=3x}}</math>] and the second is five things [<math>\scriptstyle{\color{blue}{b=5x}}</math>] | ||
| + | |style="text-align:right;"|תניח שהמספר ראשון יהיה ג' דברי' והשני יהיה ה' דברי‫' | ||
| + | |- | ||
| + | | | ||
| + | :Now, multiply the first, which is 3 things, by itself; the result is 9 squares. | ||
| + | |style="text-align:right;"|עתה תכה הראשון בעצמו שהוא ג' דברי' ויעלה ט' צינסי | ||
| + | |- | ||
| + | | | ||
| + | :Multiply 8 squares by the second number, which is 5 things; the result is 45 cubes. Keep them for one side of the equation. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(3x\right)^2\sdot5x=9x^2\sdot5x=45x^3}}</math> | ||
| + | |style="text-align:right;"|ואלו <s>הצ</s> הט' צינצי תכה במספר השני שהוא ה' דברי' ועולה מ"ה מעוק' ושמור זה בעד חלק אחד מההשואו‫' | ||
| + | |- | ||
| + | | | ||
| + | :Then, multiply the second number, which is 5 things, by itself; the result is 25 squares. | ||
| + | |style="text-align:right;"|אח"כ תכה המספר השני שהוא ה' דברי' בעצמו ועולה כ"ה צינסי | ||
| + | |- | ||
| + | | | ||
| + | :Multiply this product, i.e. 25 squares, by a root of 8: | ||
| + | |style="text-align:right;"|אח"כ תכה זאת ההכאה רצוני כ"ה צינסי בשרש ‫<ref>35r</ref>ח‫' | ||
| + | |- | ||
| + | | | ||
| + | :Remember that you must convert 25 squares into a root; you get a root of 625 squares of squares. | ||
| + | |style="text-align:right;"|ותזכור כי הנך צריך להשיב כ"ה צינצי אל שרש ויהיה לך שרש מתרכ"ה צינסי מצינסי | ||
| + | |- | ||
| + | | | ||
| + | :Multiply it by a root of 8; the result is a root of 5000 squares of squares that is equal to 45 cubes. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(5x\right)^2\sdot\sqrt{8}=25x^2\sdot\sqrt{8}=\sqrt{625x^4\sdot8}=\sqrt{5000x^4}=45x^3}}</math> | ||
| + | |style="text-align:right;"|ותכם בשרש ח' ועולה שרש ה' אלפי' צינסי מצינסי שהם שוים אל מ"ה מעוקבי‫' | ||
| + | |- | ||
| + | | | ||
| + | :Pursue the above mentioned rule: | ||
| + | |style="text-align:right;"|עתה תרדוף כפי הכלל האמור למעלה | ||
| + | |- | ||
| + | | | ||
| + | :Multiply the number of the cubes, which is 45 cubes, by itself; the result is 2025. | ||
| + | |style="text-align:right;"|תכה כמות המעוק' בעצמם וזהו מ"ה מעו' ועולה אלפיים וכ"ה | ||
| + | |- | ||
| + | | | ||
| + | :Divide the number of the squares of squares that are the radicand, which is 5000, by the 2025; the result is 2 integers and 38 [parts] of 81. | ||
| + | |style="text-align:right;"|ותחלק כמות הצינסי מצינסי הנקובי' להיות להם שרש והם ה' אלפים על אלו אלפיים וכ"ה ויבא מזה ב' שלמים ול"ח מפ"א | ||
| + | |- | ||
| + | | | ||
| + | :The root of 2 and 38 [parts] of 81 is equal to the thing. | ||
| + | :<math>\scriptstyle{\color{blue}{x=\sqrt{\frac{5000}{45^2}}=\sqrt{\frac{5000}{2025}}=\sqrt{2+\frac{38}{81}}}}</math> | ||
| + | |style="text-align:right;"|ושרש אלו הב' ול"ח מפ"א יבא לשוות הדבר | ||
| + | |- | ||
| + | | | ||
| + | :You assumed the first number is 3 things, so multiply 3 by a root of 2 and 38 [parts] of 81; the result is a root of 22 and 2-ninths and this is the first number. | ||
| + | :<math>\scriptstyle{\color{blue}{a=3x=3\sdot\sqrt{2+\frac{38}{81}}=\sqrt{22+\frac{2}{9}}}}</math> | ||
| + | |style="text-align:right;"|ואתה הנחת היות המספר הראשון ג' דברי‫'<br> | ||
| + | א"כ תכה ג' בשרש ב' ול"ח מפ"א ועולה שרש כ"ב וב' תשיעיות וככה יבא להיות המספר הראשון | ||
| + | |- | ||
| + | | | ||
| + | :You assumed the second is 5 things, so multiply 5 by a root of 2 and 38 [parts] of 81; the result is a root of 61 and 59 [parts] of 81 and this is the second number. | ||
| + | :<math>\scriptstyle{\color{blue}{b=5x=5\sdot\sqrt{2+\frac{38}{81}}=\sqrt{61+\frac{59}{81}}}}</math> | ||
| + | |style="text-align:right;"|והשני הנחת היותו ה' דברי‫'<br> | ||
| + | א"כ תכה ה' בשרש ב' ול"ח מפ"א ועולה שרש מס"א ונ"ט מפ"א וככה יבא להיות המספר השני | ||
| + | |- | ||
| + | |Know that if you wish to return it to one of the other chapters, multiply each side [of the equation] by itself; the squares of squares become equal to cubes of cubes, or squares of squares of squares. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\left(bx^3\right)^2=\left(\sqrt{ax^4}\right)^2\;\longrightarrow\;ax^4=Bx^6}}</math> | ||
| + | |style="text-align:right;"|<big>ודע</big> שאם רצית להשיבה אל פרק מה מהאחרי' תכה עתה כל אחד מן החלקי' בעצמו והיה עולה צינסי מצינסי אל מעוק' המעוק' או שוה אל {{#annot:term|689|hdFg}}צינסו מצינסו מצינסו{{#annotend:hdFg}} | ||
| + | |- | ||
| + | |Divide this equation by a square; it becomes squares equal squares of squares and it is returned to chapter 13. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{ax^4=Bx^6\;/\div x^2\;\longrightarrow\;ax^2=Bx^4}}</math> | ||
| + | |style="text-align:right;"|וזאת ההשואה בחלוק [אותה]‫<ref>marg.</ref> על צינסי יעלה יבא צינסי שוה אל צינסו מצינסו והיתה מושבת אל הפרק הי"ג | ||
| + | |- | ||
| + | |Dividing it by a cube; it becomes things equal cubes and it is returned to chapter 8. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{ax^4=Bx^6\;/\div x^3\;\longrightarrow\;ax=Bx^3}}</math> | ||
| + | |style="text-align:right;"|ובחלקה על מעו' יבא דבר שוה אל מעו' והיתה מושבת אל פרק ח‫' | ||
| + | |- | ||
| + | |Dividing it by a square of a square, it is returned to the second chapter, which is numbers equal squares. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{ax^4=Bx^6\;/\div x^4\;\longrightarrow\;a=Bx^2}}</math> | ||
| + | |style="text-align:right;"|ובחלקה על צינסי מצינסו היתה באה לך <s>אל</s> מושבת אל הפרק השני שהוא מספר שוה אל צינסו | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== Chapter 35 ==== | ||
| + | |||
| + | |style="text-align:right;"|<big>פרק ל"ה</big> | ||
| + | |- | ||
| + | |When the squares of squares are equal to [a root of] squares of squares: | ||
| + | :<math>\scriptstyle ax^4=\sqrt{bx^4}</math> | ||
| + | |style="text-align:right;"|עוד באופן אחר דהיינו כאשר הצינסי מצינסי הם שוים אל צינסי מצינסי | ||
| + | |- | ||
| + | |The number of squares of squares should be multiplied by itself. | ||
| + | |style="text-align:right;"|צריך להכות כמות הצינסי מצינסי בעצמם | ||
| + | |- | ||
| + | |Then, the number of squares of squares that are the radicand should be divided by this product. | ||
| + | |style="text-align:right;"|ולחלק כמות הצינסי מצינסי הנקובי' להיות להם שרש על ההכאה ההיא | ||
| + | |- | ||
| + | |The root of a root of the result is equal to the things. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{x=\sqrt[4]{\frac{b}{a^2}}}}</math> | ||
| + | |style="text-align:right;"|ושרש שרש מהעולה יבא לשוות הדבר | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:a/b=⁵/₇;(ab)²=a²√8|618|3fAj}}Find me two numbers such that the one is a part of the other as 5 is of 7; | ||
| + | :and if the first is multiplied the second, then the product is multiplied by itself, it yields the same as the product of the smaller by itself, then the product multiplied by the root of 8. | ||
| + | :I ask: how much will each number be? | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle a:b=5:7\\\scriptstyle\left(a\sdot b\right)^2=a^2\sdot\sqrt{8}\end{cases}</math> | ||
| + | |style="text-align:right;"|תמצא לי שני מספרי' שיהיה האחד חלק מהאחר כאשר ה' הוא מז‫'<br> | ||
| + | ומוכה הראשון בשני ואותה ההכאה תוכה בעצמה יעשה כמו הכאת המספר הקטן בעצמו וההכאה ההיא תוכה בשרש ח‫'<br> | ||
| + | אשאל כמה יבא להיות כל אחד מהמספרי‫'{{#annotend:3fAj}} | ||
| + | |- | ||
| + | | | ||
| + | :This is its rule: | ||
| + | |style="text-align:right;"|זהו כללו | ||
| + | |- | ||
| + | | | ||
| + | :Suppose the first number is five things [<math>\scriptstyle{\color{blue}{a=5x}}</math>] and the other is seven things [<math>\scriptstyle{\color{blue}{b=7x}}</math>] | ||
| + | |style="text-align:right;"|תניח שהמספר הראשון יהיה ה' דברי' והאחר ז' דברי‫' | ||
| + | |- | ||
| + | | | ||
| + | :Now, multiply the first by the second, which is 5 things by 7 things; the result is 35 squares. | ||
| + | |style="text-align:right;"|עתה תכה הראשון בשני וזהו ה' דברי' בז' דברי' ועולה ל"ה צינסי | ||
| + | |- | ||
| + | | | ||
| + | :Then, multiply this product, i.e. 35 squares, by itself; the result is 1225 squares of squares. Keep them for one side of the equation. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(5x\sdot7x\right)^2=\left(35x^2\right)^2=1225x^4}}</math> | ||
| + | |style="text-align:right;"|אח"כ תכה זאת ההכאה רצוני ל"ה צינסי בעצמם ועולה אלף ורכ"ה צינסי מצינסי ושמור בעד אחד מחלקי ההשואה | ||
| + | |- | ||
| + | | | ||
| + | :Multiply the smaller number, which is 5 things, by itself; the result is 25 squares. | ||
| + | |style="text-align:right;"|אח"כ תכה המספר הקטו' שהוא ה' דברי' בעצמו ועולה כ"ה צינסי | ||
| + | |- | ||
| + | | | ||
| + | :Multiply the 25 squares by a root of 8: | ||
| + | |style="text-align:right;"|ותכה אלו הכ"ה צינסי בשרש ח‫' | ||
| + | |- | ||
| + | | | ||
| + | :Remember that you must convert 25 squares into a root; you get a root of 625 squares of squares. | ||
| + | |style="text-align:right;"|וזכור כי הנך צריך להשיב כ"ה צינסי אל שרש ויהיה לך שרש מתרכ"ה צינסי מצינסי | ||
| + | |- | ||
| + | | | ||
| + | :Multiply it by a root of 8; the result is a root of 5000 squares of squares that is equal to 1225 squares of squares. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(5x\right)^2\sdot\sqrt{8}=25x^2\sdot\sqrt{8}=\sqrt{625x^4\sdot8}=\sqrt{5000x^4}=1225x^4}}</math> | ||
| + | |style="text-align:right;"|ותכם בשרש ח' ועולה שרש ה' אלפי' צינסי מצינסי שהם שוים אל אלף ורכ"ה צינסי מצינסי | ||
| + | |- | ||
| + | | | ||
| + | :Pursue the above said rule: | ||
| + | |style="text-align:right;"|עתה תרדוף כפי הכלל האמור למעלה | ||
| + | |- | ||
| + | | | ||
| + | :Multiply the number of the squares of squares, which is 1225, by itself; the result is 1500625. | ||
| + | |style="text-align:right;"|תכה כמות הצינסי בצינסי בעצמם והם אלף ורכ"ה ועולה אלף אלפי' ות"ק אלפי' ותרכ"ה | ||
| + | |- | ||
| + | | | ||
| + | :Divide the number of squares of squares that are the radicand of the equation, which is 5000, by 1500625; the result is 8 parts of 2401. | ||
| + | |style="text-align:right;"|ותחלק כמות הצינסי מצינסי הנקובי' להיות להם שרש בהשואה שהם ה' אלפי' על אלף אלפים ות"ק אלפי' ותרכ"ה ויבא מזה ח' חלקי' מאלפיים ות"א | ||
| + | |- | ||
| + | | | ||
| + | :Extract the root of the root of the 8 parts of 2401 and this is equal to the thing. | ||
| + | :<math>\scriptstyle{\color{blue}{x=\sqrt[4]{\frac{5000}{1225^2}}=\sqrt[4]{\frac{5000}{1500625}}=\sqrt[4]{\frac{8}{2401}}}}</math> | ||
| + | |style="text-align:right;"|ותקח שרש השרש מאלו ‫<ref>35v</ref>הח' חלקי' מאלפיים ות"א וככה יבא לשוות הדבר | ||
| + | |- | ||
| + | | | ||
| + | :You assumed the first number is 5 things, so multiply 5 by a root of a root of 8 parts of 2401; the result is a root of a root of 2 and 198 parts of 2401 and this is the first number. | ||
| + | :<math>\scriptstyle{\color{blue}{a=5x=5\sdot\sqrt[4]{\frac{8}{2401}}=\sqrt[4]{2+\frac{198}{2401}}}}</math> | ||
| + | |style="text-align:right;"|ואתה הנחת היות המספר ראשון ה' דברי‫'<br> | ||
| + | א"כ תכה ה' בשרש שרש מח' חלקי' מאלפיים ת"א ועולה שרש משרש ב' וקצ"ח חלקי' מאלפיים ות"א וככה יבא להיות המספר ראשון | ||
| + | |- | ||
| + | | | ||
| + | :You assumed the second is 7 things, so multiply 7 by a root of a root of 8 parts of 2401; the result is a root of a root of 8 and this is the second number. | ||
| + | :<math>\scriptstyle{\color{blue}{b=7x=7\sdot\sqrt[4]{\frac{8}{2401}}=\sqrt[4]{8}}}</math> | ||
| + | |style="text-align:right;"|והשני הנחת היותו ז' דברי‫'<br> | ||
| + | א"כ תכה ז' בשרש שרש מח' חלקי' מאלפיים ות"א ועולה שרש משרש ח' וככה יבא להיות המספר השני | ||
| + | |- | ||
| + | |Know that this calculation can be returned to chapter 11, by multiplying each side of the equation by itself: | ||
| + | |style="text-align:right;"|<big>ודע</big> כי זה החשבון אפשר להשיבו אל הפרק הי"א בזה האופן בהכות כל אחד מחלקי ההשואה בעצמו | ||
| + | |- | ||
| + | |The root of squares of squares becomes squares of squares. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\left(\sqrt{bx^4}\right)^2=bx^4}}</math> | ||
| + | |style="text-align:right;"|ושרש צינסו מצינסו יבא צינסו מצינסו | ||
| + | |- | ||
| + | |The squares of squares become squares of squares of squares of squares. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\left(ax^4\right)^2=Ax^8}}</math> | ||
| + | |style="text-align:right;"|והצינסי מצינסי יבא {{#annot:term|689|yHS1}}צינסו מצינסו מצינסו מצינסו{{#annotend:yHS1}} | ||
| + | |- | ||
| + | |Then, dividing it by squares of squares: | ||
| + | |style="text-align:right;"|ואח"כ בחלוק זה על צינסו מצינסו | ||
| + | |- | ||
| + | |The squares of squares become numbers. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{bx^4\div x^4=b}}</math> | ||
| + | |style="text-align:right;"|{{#annot:term|875,1566|nGmC}}יבאו לך ה{{#annotend:nGmC}}צינסי מצינסי מספרי‫' | ||
| + | |- | ||
| + | |And the squares of squares of squares of squares become squares of squares. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{Ax^8\div x^4=Ax^4}}</math> | ||
| + | |style="text-align:right;"|וה{{#annot:term|689|RcAh}}צינסי מצינסי מצינסי מצינסי{{#annotend:RcAh}} יבאו צינסי מצינסי | ||
| + | |- | ||
| + | |So, it is returned to the said chapter 11, as we intended to return it. | ||
| + | |style="text-align:right;"|והיה מושב אל הפרק <sup>הי"א</sup> האמור <s>הי"א</s> כמו שאמרנו להשיבו | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== Chapter 36 ==== | ||
| + | |||
| + | |style="text-align:right;"|<big>פרק ל"ו</big> | ||
| + | |- | ||
| + | |When things are equal to numbers and a root of numbers: | ||
| + | :<math>\scriptstyle bx=c+\sqrt{d}</math> | ||
| + | |style="text-align:right;"|כאשר הדברי' יהיו שוים אל מספרי' ואל שרשי מספרי‫' | ||
| + | |- | ||
| + | |The numbers that are [not] the radicand should be divided by the number of the things, and the result is kept. | ||
| + | |style="text-align:right;"|צריך לחלק כמות המספרי' הנקוב היות לו שרש <sup>על</sup><s>ב</s>כמות הדברים ולשמור העולה | ||
| + | |- | ||
| + | |Then, the number of the things should be multiplied by itself. | ||
| + | |style="text-align:right;"|ואח"כ להכות כמות הדברי' בעצמם | ||
| + | |- | ||
| + | |The number that is the radicand should be divided by this product. | ||
| + | |style="text-align:right;"|ולחלק המספרי' הנקובי' להיות להם שרש על אותה הכאה | ||
| + | |- | ||
| + | |Add the root of the result to the quotient we said should be kept; the result is equal to the thing and it is a number and a root. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{x=\frac{c}{b}+\sqrt{\frac{d}{b^2}}}}</math> | ||
| + | |style="text-align:right;"|ושרש העולה מזה תחבר אל מה שעלה מהחלוק שאמרנו לשמרו ומה שיעלה ישוה הדבר ויהיה מספר ושרש | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:a/b=⅔;5a+7b=16+√8|618|qQCL}}Find me two numbers such that the one is a part of the other as 2 is of 3; | ||
| + | :and when the first is multiplied by 5 and the second by 7, then both the products are summed together, it yields 16 and a root of 8. | ||
| + | :I ask: how much will each number be? | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle a:b=2:3\\\scriptstyle5a+7b=16+\sqrt{8}\end{cases}</math> | ||
| + | |style="text-align:right;"|תמצא לי שני מספרי' שיהיה האחד מהאחר כמו שב' הוא מג‫'<br> | ||
| + | ומוכה הראשון בה' והשני בז' ושתי אלו ההכאות יחוברו יחד יעשו י"ו ושרש ח‫'<br> | ||
| + | אשאל כמו' יבא להיות כל אחד מהמספרי‫'{{#annotend:qQCL}} | ||
| + | |- | ||
| + | | | ||
| + | :This is the calculation procedure: | ||
| + | |style="text-align:right;"|זאת היא {{#annot:term|469|GL9Q}}פעלת{{#annotend:GL9Q}} זה החשבון | ||
| + | |- | ||
| + | | | ||
| + | :Suppose the first number is two things [<math>\scriptstyle{\color{blue}{a=2x}}</math>] and the second is three things [<math>\scriptstyle{\color{blue}{b=3x}}</math>]. | ||
| + | |style="text-align:right;"|תניח שהמספר ראשון יהיה ב' דברי' והשני ג' דברי‫' | ||
| + | |- | ||
| + | | | ||
| + | :Now, multiply the first, which is 2 things, by 5; the result is ten things. | ||
| + | :<math>\scriptstyle{\color{blue}{5\sdot2x=10x}}</math> | ||
| + | |style="text-align:right;"|עתה תכה הראשון שהוא ב' דברי' בה' ויעלה עשרה דברי‫' | ||
| + | |- | ||
| + | | | ||
| + | :Then, multiply the second number, which is 3 things, by 7; the result is 21 things. | ||
| + | :<math>\scriptstyle{\color{blue}{7\sdot3x=21x}}</math> | ||
| + | |style="text-align:right;"|אח"כ תכה המספר השני שהוא ג' דברי' בז' ועולה כ"א דברי‫' | ||
| + | |- | ||
| + | | | ||
| + | :Add them to the first product, which is 10 things; you get 31 things. Keep them for one side [of the equation]. | ||
| + | :<math>\scriptstyle{\color{blue}{10x+21x=31x}}</math> | ||
| + | |style="text-align:right;"|וחברם עם ההכאה הראשונה שהיא י' דברי' ויהיה לך ל"א דברי' ושמרם בעד המנגד מהחלק האחד | ||
| + | |- | ||
| + | | | ||
| + | :You have 31 things equal 16 and a root of 8. | ||
| + | :<math>\scriptstyle31x=16+\sqrt{8}</math> | ||
| + | |style="text-align:right;"|ויהיה לך ל"א דברי' שוים אל י"ו ושרש ח‫' | ||
| + | |- | ||
| + | | | ||
| + | :Pursue the above mentioned rule: | ||
| + | |style="text-align:right;"|עתה תרדוף כפי הכלל האמור למעלה | ||
| + | |- | ||
| + | | | ||
| + | :Divide the number by the number of the things, it is 16 by 31; the result is 16 [parts] of 31. Keep it. | ||
| + | |style="text-align:right;"|תחלק המספרי' בכמות הדברי' וזהו י"ו על ל"א אשר יעלה מזה י"ו מל"א ושמור זה | ||
| + | |- | ||
| + | | | ||
| + | :Then, convert the number of the things, which is 31, into a root; the result is 961. | ||
| + | |style="text-align:right;"|אח"כ תשיב כמות הדברי' אל שרש שהם ל"א ועולה תתקס"א | ||
| + | |- | ||
| + | | | ||
| + | :Divide the number that is the radicand, which is 8, by 961; the result is 8 [parts] of 961 and this is the thing. | ||
| + | :<math>\scriptstyle{\color{blue}{x=\frac{16}{31}+\sqrt{\frac{8}{31^2}}=\frac{16}{31}+\sqrt{\frac{8}{961}}}}</math> | ||
| + | |style="text-align:right;"|ותחלק המספרי' הנקובי' להיות להם שרש שהם ח' על תתקס"א ועולה מזה ח' מתתקס"א וככה שוה הדבר | ||
| + | |- | ||
| + | | | ||
| + | :You assumed the first number is 2 things, so multiply 2 by 16 [parts] of 31 and a root of 8 [parts] of 961; the result is 1 and one [part] of 31 and a root of 32 [parts] of 961; this is the first number. | ||
| + | :<math>\scriptstyle{\color{blue}{a=2x=2\sdot\left(\frac{16}{31}+\sqrt{\frac{8}{961}}\right)=1+\frac{1}{31}+\sqrt{\frac{32}{961}}}}</math> | ||
| + | |style="text-align:right;"|ואתה הנחת היות המספר ראשון ב' דברי‫'<br> | ||
| + | א"כ תכה ב' בי"ו מל"א ושרש ח' מתתקס"א ועולה א' וא' מל"א ושרש ל"ב מתתקס"א וככה יבא להיות המספר ראשון | ||
| + | |- | ||
| + | | | ||
| + | :You assumed the second is 3 things, so multiply 3 by 16 [parts] of 31 and a root of 8 [parts] of 961; the result is 1 and 17 [parts] of 31 and a root of 72 [parts] of 961; this is the second number. | ||
| + | :<math>\scriptstyle{\color{blue}{b=3x=3\sdot\left(\frac{16}{31}+\sqrt{\frac{8}{961}}\right)=1+\frac{17}{31}+\sqrt{\frac{72}{961}}}}</math> | ||
| + | |style="text-align:right;"|והשני הנחת היותו ג' דברי‫'<br> | ||
| + | א"כ תכה ג' בי"ו מל"א ושרש ח' מתתקס"א ועולה א' וי"ז מל"א ושרש ע"ב מתתקס"א וככה הוא המספר השני | ||
| + | |- | ||
| + | |This calculation is done according the the rule of [the present] chapter, as well as] the first chapter and [chapter] 18. | ||
| + | |style="text-align:right;"|ויבא להיות נעשה זה החשבון מן הכלל [הפרק]<ref>marg.</ref> הראשון והי"ח | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== Chapter 37 ==== | ||
| + | |||
| + | |style="text-align:right;"|<big>הפרק הל"ז</big> | ||
| + | |- | ||
| + | |When numbers are equal to things and a root of things: | ||
| + | :<math>\scriptstyle c=ax+\sqrt{bx}</math> | ||
| + | |style="text-align:right;"|כאשר המספרי' הם שוים אל דברי' ושרשי דברי‫' | ||
| + | |- | ||
| + | |The numbers should be divided by the number of the things that are not the radicand. | ||
| + | |style="text-align:right;"|צריך לחלק המספרי' בכמות הדברי' שאינם נקובי' להיות להם ‫<ref>36r</ref>שרש | ||
| + | |- | ||
| + | |Then, the number of the things that are [not] the radicand should be multiplied by itself. | ||
| + | |style="text-align:right;"|ואח"כ להכות כמות הדברי' הנקובי' להיות להם שרש בעצמם | ||
| + | |- | ||
| + | |The number of the things that are the radicand should be divided by this product. | ||
| + | |style="text-align:right;"|ולחלק כמות הדברי' הנקובי' להיות להם שרש על אותה ההכאה | ||
| + | |- | ||
| + | |Add a quarter of the result to the quotient of the numbers divided by the number of the things. | ||
| + | |style="text-align:right;"|ורביע מהעולה תחבר על {{#annot:term|783,1566|wZdW}}המספר הבא בחלוק{{#annotend:wZdW}} המספר על כמות הדברי‫' | ||
| + | |- | ||
| + | |When the root of the quarter that is added to the number - i.e. the quotient of the number of the things that are the radicand divided by the product of the number of the things that are not the radicand - is subtracted from the root of the sum, the result is the root of the thing. | ||
| + | |style="text-align:right;"|ושרש זה הסך בהיות מוצא שרש מהרביע אשר חובר אל המספר דהיינו מ{{#annot:term|783,1223|C2SF}}החלוק הבא לך בחלוק{{#annotend:C2SF}} כמות הדברי' הנקובי' להיות להם שרש על הכאת כמות הדברי' הבלתי נקובי' להיות להם שרש יבא להיות שרש הדבר | ||
| + | |- | ||
| + | |When it is multiplied by itself, the result is equal to the thing. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{x=\left(\sqrt{\frac{c}{a}+\left(\frac{1}{4}\sdot\frac{b}{a^2}\right)}-\sqrt{\frac{1}{4}\sdot\frac{b}{a^2}}\right)^2}}</math> | ||
| + | |style="text-align:right;"|ובהכות זה על עצמו יבא לשוות הדבר | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:a/b=⅔;3a+4√b=30|618|37bI}}Find me two numbers such that the one is a part of the other as 2 is of 3; | ||
| + | :and when the first is multiplied by 3 and the root of the second by 4, then both the products are summed together, it yields thirty. | ||
| + | :I ask: how much will each number be? | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle a:b=2:3\\\scriptstyle3a+4\sqrt{b}=30\end{cases}</math> | ||
| + | |style="text-align:right;"|והנה המשל <big>תמצא</big> לי שני מספרי' שיהיה האחד חלק מאחר כמו שב' הוא מג‫'<br> | ||
| + | ובהכות הראשון בג' ושרש השני בד' ויחוברו שתי אלו ההכאות יחד יעשה שלשים<br> | ||
| + | אשאל כמה יבא להיות כל אחד מהמספרי‫'{{#annotend:37bI}} | ||
| + | |- | ||
| + | |The procedure: | ||
| + | |style="text-align:right;"|זהו {{#annot:term|469,1427|TvqK}}מעשהו{{#annotend:TvqK}} | ||
| + | |- | ||
| + | | | ||
| + | :Suppose the first number is two things [<math>\scriptstyle{\color{blue}{a=2x}}</math>] and the other is three things [<math>\scriptstyle{\color{blue}{b=3x}}</math>] | ||
| + | |style="text-align:right;"|תניח שהמספר ראשון יהיה ב' דברי' והאחר יהיה ג' דברים | ||
| + | |- | ||
| + | | | ||
| + | :<math>\scriptstyle{\color{blue}{3\sdot2x=6x}}</math> | ||
| + | |style="text-align:right;"|עתה תכה הראשון שהוא ב' דברי' בג' עולה ו' דברי' ושמרם | ||
| + | |- | ||
| + | | | ||
| + | :<math>\scriptstyle{\color{blue}{4\sdot\sqrt{3x}=\sqrt{16\sdot3x}=\sqrt{48x}}}</math> | ||
| + | |style="text-align:right;"|אח"כ תכה שרש השני שזה הוא שרש מג' דברי' בד‫' | ||
| + | |- | ||
| + | | | ||
| + | :Remember that you must convert 4 into a root; you get a root of 16. | ||
| + | |style="text-align:right;"|ותזכור כי הנך צריך להשיב ד' אל שרש ויהיה לך שרש מי"ו<br> | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ותכה זה בשרש ג' דברי' ועולה שרש מ"ח דברי‫' | ||
| + | |- | ||
| + | | | ||
| + | :summing both products together: | ||
| + | |style="text-align:right;"|ותחבר שתי אלו ההכאות יחד | ||
| + | |- | ||
| + | | | ||
| + | :<math>\scriptstyle{\color{blue}{6x+\sqrt{48x}}}</math> | ||
| + | |style="text-align:right;"|וזהו ו' דברי' עם שרש מ"ח דברי‫' | ||
| + | |- | ||
| + | | | ||
| + | :<math>\scriptstyle6x+\sqrt{48x}=30</math> | ||
| + | |style="text-align:right;"|ויהיה לך ו' דברי' ושרש ממ"ח דברי' והם באים להיות שוים אל ל‫' | ||
| + | |- | ||
| + | | | ||
| + | :Pursue the above said rule: | ||
| + | |style="text-align:right;"|עתה תרדוף כפי הכלל האמור למעלה | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|תחלק המספרי' בכמות הדברי' הנקובי' להיות להם שרש והם ל' על ו' ועולה מזה ה' ושמרהו | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|אח"כ תכה כמות הדברי' אשר לא נוקבו להיות להם שרש שהם ו' בעצמם ועולה ל"ו | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ותחלק כמות הדברי' הנקובי' להיות להם שרש שהם מ"ח על ל"ו ויבא מזה א' ושליש | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ותחבר הרביע מא' ושליש עם המספר אשר שמרת והוא על ה' ויהיה לך ה' ורביע | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ושרש זה הסך פחות שרש א' רביע אשר בא לנו בחלוק כמות הדברי' אשר <s>לא</s> נקבו להיות להם שרש על הכאת כמות הדברי' אשר לא נקבו להיות להם שרש שזהו פחות שרש שליש יבא להיות שרש הדבר | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|והוא מוצא שרש א' שליש מהשרש מחבור ה' וא' שליש וזהו ה' ושליש יבא להיות שרש הדבר האמור | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ואותו תכפול בעצמו באופן זה באמור שרש ה' וא' שליש פחות שרש מא' שליש מוכה בשרש ה‫' וא' שליש פחות שרש א' שליש שעולה <sup>ב</sup>ה' וב' שלישי פחות שרש מס"ד תשיעיות | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|אשר זה השרש הוא ב' וב' שלישי | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ותוציאהו מה' וב' שלישי וישאר ג' וככה <s>הוא</s> שוה הדבר | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle x&\scriptstyle=\left(\sqrt{\frac{30}{6}+\left(\frac{1}{4}\sdot\frac{48}{6^2}\right)}-\sqrt{\frac{1}{4}\sdot\frac{48}{6^2}}\right)^2=\left(\sqrt{5+\left(\frac{1}{4}\sdot\frac{48}{36}\right)}-\sqrt{\frac{1}{4}\sdot\frac{48}{36}}\right)^2=\left[\sqrt{5+\left[\frac{1}{4}\sdot\left(1+\frac{1}{3}\right)\right]}-\sqrt{\frac{1}{4}\sdot\left(1+\frac{1}{3}\right)}\right]^2\\&\scriptstyle=\left(\sqrt{5+\frac{1}{3}}-\sqrt{\frac{1}{3}}\right)^2=5+\frac{2}{3}-\sqrt{\frac{64}{9}}=5+\frac{2}{3}-\left(2+\frac{2}{3}\right)=3\\\end{align}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :<math>\scriptstyle{\color{blue}{a=2x=2\sdot3=6}}</math> | ||
| + | |style="text-align:right;"|ואתה הנחת היות המספר ראשון ב' דברי‫'<br> | ||
| + | א"כ תכה ג' בב' עולה ו' וככה יבא להיות המספר ראשון | ||
| + | |- | ||
| + | | | ||
| + | :<math>\scriptstyle{\color{blue}{b=3x=3\sdot3}}</math> | ||
| + | |style="text-align:right;"|ואתה הנחת שהשני היה ג' דברי‫'<br> | ||
| + | והדבר יבא להיות ג' א"כ תכה ג' בג' וככה יבא להיות המספר השני | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== Chapter 38 ==== | ||
| + | |||
| + | |style="text-align:right;"|<big>פרק ל"ח</big> | ||
| + | |- | ||
| + | |When squares are equal to numbers and a root of numbers: | ||
| + | :<math>\scriptstyle ax^2=c+\sqrt{d}</math> | ||
| + | |style="text-align:right;"|כאשר הצינסי יהיו שוים אל מספרי' ולשרשי מספרי‫' | ||
| + | |- | ||
| + | |The numbers should be divided by the number of the squares. | ||
| + | |style="text-align:right;"|צריך לחלק המספר על כמות הצינסי והעולה תשמור | ||
| + | |- | ||
| + | |Then, the number of the squares should be multiplied by itself. | ||
| + | |style="text-align:right;"|ואח"כ להכות כמות הצינסי בעצמם | ||
| + | |- | ||
| + | |The numbers that are the radicand should be divided by this product. | ||
| + | |style="text-align:right;"|ולחלק המספרי' אשר נקבו להיות להם שרש על אותה ההכאה | ||
| + | |- | ||
| + | |The root of the quotient is added to the number you kept, i.e. the result of division of the numbers by the number of the squares; the sum is equal to the square. | ||
| + | |style="text-align:right;"|ושרש חלק מה שיעלה מחובר עם המספר אשר שמרת דהיינו מאותו שעלה לך בחלוק המספרי' על כמות הצינסי ומה שיעלה הסך ישוה הצינסו | ||
| + | |- | ||
| + | |The root of the mentioned sum is equal to the thing. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{x=\sqrt{\frac{c}{a}+\sqrt{\frac{d}{a^2}}}}}</math> | ||
| + | |style="text-align:right;"|ושרש הסך האמור ישוה הדבר | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:a/b=⅔;ab=20+√8|618|B6TE}}Find me two numbers such that the first is a part of the second as 2 is of 3; | ||
| + | :and when the first is multiplied by the second it yields 20 and a root of 8. | ||
| + | :I ask: how much will each number be? | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle a:b=2:3\\\scriptstyle a\sdot b=20+\sqrt{8}\end{cases}</math> | ||
| + | |style="text-align:right;"|תמצא לי שני מספרים שיהיה הראשון חלק מהשני כמו שב' הוא מג‫'<br> | ||
| + | ומוכה הראשון בשני יעשה כ' ושרש ח‫'<br> | ||
| + | אשאל כמה יבא להיות כל אחד מהמספרי‫'{{#annotend:B6TE}} | ||
| + | |- | ||
| + | |The procedure: | ||
| + | |style="text-align:right;"|זהו מעשהו | ||
| + | |- | ||
| + | | | ||
| + | :defining: | ||
| + | :*the first number as two things <math>\scriptstyle{\color{blue}{a=2x}}</math> | ||
| + | |style="text-align:right;"|תניח שהמספר ראשון יהיה ב' דברים | ||
| + | |- | ||
| + | | | ||
| + | :*the second will be three things <math>\scriptstyle{\color{blue}{b=3x}}</math> | ||
| + | |style="text-align:right;"|והשני יהיה ג' דברי‫' | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{2x\sdot3x}}</math> | ||
| + | |style="text-align:right;"|עתה תכה הראשון בשני וזהו ב' דברים בג' דברי‫' | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle6x^2=20+\sqrt{8}</math> | ||
| + | |style="text-align:right;"|ויהיו ו' צינסי שהם שוים אל כ' ושרש ח‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Pursuing the above rule: | ||
| + | |style="text-align:right;"|עתה תרדוף כפי הכלל הנתון למעלה | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|תחלק המספרים אשר לא נקבו להיות להם שרש והם כ' על כמות הצינסי שהם ו' שעולה מזה ג' וא' צינסו ושמרם | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|אח"כ תכה כמות הצינסי בעצמם והם ו' ועולה ל"ו | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ותחלק המספרי' הנקובי' להיות להם שרש שזהו ח' על ל"ו שעולה מזה ב' תשיעיות | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ושרש אלו הב' תשיעיות תחבר עם אלו הג' וא' שליש שהוא מה שבא מהחלוק מהמספרי' על כמות הצינסי<br> | ||
| + | ויהיה לך שרש מחבור ג' ושליש עם שרש ב' תשיעיו' וככה ישוה הדבר | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{x=\sqrt{\frac{20}{6}+\sqrt{\frac{8}{6^2}}}=\sqrt{\left(3+\frac{1}{3}\right)+\sqrt{\frac{8}{36}}}=\sqrt{\left(3+\frac{1}{3}\right)+\sqrt{\frac{2}{9}}}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ואתה הנחת שהמספר ראשון היה ב' דברים<br> | ||
| + | א"כ תכה ב' בשרש חבור ג' ושליש עם שרש ב' תשיעיו' ועולה שרש מחבור י"ג ושליש עם שרש ג' וה' תשיעיו' וככה יבא להיות המספר הראשון | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{a=2x=2\sdot\sqrt{\left(3+\frac{1}{3}\right)+\sqrt{\frac{2}{9}}}=\sqrt{\left(13+\frac{1}{3}\right)+\sqrt{3+\frac{5}{9}}}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|והשני הנחת היותו ג' דברים<br> | ||
| + | א"כ תכה ג' בשרש חבור ג' ושליש עם שרש ב' תשיעיו' ועולה שרש מחבור ל' עם שרש מי"ח וככה יבא להיות המספר השני | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{b=3x=3\sdot\sqrt{\left(3+\frac{1}{3}\right)+\sqrt{\frac{2}{9}}}=\sqrt{30+\sqrt{18}}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== Chapter 39 ==== | ||
| + | |||
| + | |style="text-align:right;"|<big>פרק ל"ט</big> | ||
| + | |- | ||
| + | |When numbers are equal to squares and a root of squares: | ||
| + | :<math>\scriptstyle c=ax^2+\sqrt{bx^2}</math> | ||
| + | |style="text-align:right;"|עוד באופן אחר כאשר המספרי' יהיו שוים אל הצינסי ואל שרשי צינסי | ||
| + | |- | ||
| + | |The numbers should be divided by the number of the squares that are not the radicand. Keep the result. | ||
| + | |style="text-align:right;"|צריך לחלק המספרי' על כמות הצינסי אשר לא נקבו להיות להם שרש ומה שיעלה מזה תשמור | ||
| + | |- | ||
| + | |Then, multiply the number of the squares that are not the radicand by itself. | ||
| + | |style="text-align:right;"|ואח"כ תכה כמות הצינסי אשר לא נקבו להיות להם שרש בעצמם | ||
| + | |- | ||
| + | |The [number of the] squares that are the radicand should be divided by this product, which is as the square of the squares. | ||
| + | |style="text-align:right;"|ולחלק הצינסי אשר נקבו להיות להם שרש באותה ההכאה שהיא כרבוע הצינסי | ||
| + | |- | ||
| + | |Add a quarter of the result to the quotient of the numbers divided by the number of the squares. | ||
| + | |style="text-align:right;"|ורביע מהעולה מזה תחבר אל מה שעלה מחלוקת המספרי' על כמות הצינסי | ||
| + | |- | ||
| + | |The root of this sum minus the root of the quarter you added is equal to the thing, and it is a root of a number minus a root of a number. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{x=\sqrt{\frac{c}{a}+\left(\frac{1}{4}\sdot\frac{b}{a^2}\right)}-\sqrt{\frac{1}{4}\sdot\frac{b}{a^2}}}}</math> | ||
| + | |style="text-align:right;"|ושרש זה הסך פחות שרש אותו הרביע אשר חברת יבא לשוות הדבר<br> | ||
| + | ויהיה שרש מספר פחות שרש מספר | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:a/b=¾;a√8+b²=48|618|aGAJ}}Find me two numbers such that the one is a part of the other as 3 is of 4; | ||
| + | :and if the first is multiplied by the root of 8, and the second is multiplied by itself, then both products are summed together, it yields 48. | ||
| + | :I ask: how much will each number be? | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle a:b=3:4\\\scriptstyle\left(a\sdot\sqrt{8}\right)+b^2=48\end{cases}</math> | ||
| + | |style="text-align:right;"|תמצא לי שני מספרי' שהאחד יהיה חלק מהאחר כמו שג' הוא מד‫'<br> | ||
| + | ומוכה הראשון בשרש ח' והשני בעצמו ואותן שתי הכאות יחוברו יחד יעשו מ"ח<br> | ||
| + | אשאל כמה יבא להיות כל האחד מהמספרי‫'{{#annotend:aGAJ}} | ||
| + | |- | ||
| + | |The procedure: | ||
| + | |style="text-align:right;"|זה מעשהו | ||
| + | |- | ||
| + | | | ||
| + | :defining: | ||
| + | :*the first number as three things <math>\scriptstyle{\color{blue}{a=3x}}</math> | ||
| + | |style="text-align:right;"|תניח שהמספר ראשון יהיה ג' דברים | ||
| + | |- | ||
| + | | | ||
| + | :*the second as four things <math>\scriptstyle{\color{blue}{b=4x}}</math> | ||
| + | |style="text-align:right;"|והשני ד' דברי‫' | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{3x\sdot\sqrt{8}=\sqrt{72x^2}}}</math> | ||
| + | |style="text-align:right;"|עתה תכה הראשון שהוא ג' דברי' בשרש ח' ועולה שרש מע"ב צינסי | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{\left(4x\right)^2=16x^2}}</math> | ||
| + | |style="text-align:right;"|אח"כ תכה המספר השני שהוא ד' דברי' בעצמו ועולה י"ו צינסי | ||
| + | |- | ||
| + | | | ||
| + | :::summing both products together: | ||
| + | |style="text-align:right;"|וחבר שתי אלו ההכאות יחד | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{\sqrt{72x^2}+16x^2}}</math> | ||
| + | |style="text-align:right;"|שהם י"ו צינסי עם שרש ע"ב צינסי | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle16x^2+\sqrt{72x^2}=48</math> | ||
| + | |style="text-align:right;"|ויהיה לך י"ו צינסי ושרש ע"ב צינסי שוים אל מ"ח | ||
| + | |- | ||
| + | | | ||
| + | ::Pursuing the above rule: | ||
| + | |style="text-align:right;"|עתה תרדוף כפי הכלל הנתון למעלה | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|תחלק המספרים בכמות הצינסי אשר אינם נקובים להיות להם שרש שהם מ"ח בי"ו ויבא מזה ג' ושמרם | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|אח"כ תכה כמות הצינסי הבלתי נקובים להיות להם שרש בעצמם וזהו י"ו ועולה רנ"ו | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|וחלק הצינסי הנקובי' להיות להם שרש וזהו ע"ב על רנ"ו ויבא מזה ט' חלקים מל"ב<br> | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|עתה תקח הרביע מאלו הט' חלקים מל"ב ויהיה לך חלקים מקכ"ח | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ואלו הט' מקכ"ח תחבר על המספר שהוא ג' אשר בא {{#annot:term|784,1259|nCpc}}בחלקך{{#annotend:nCpc}} המספרי' על הצינסי והוא אשר שמרת ויהיה לך ג' וט' מקכ"ח | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ושרש ג' וט' מקכ"ח פחות שרש מאלו הט' מקכ"ח שהוא הרביע ממה שבא אליך בחלקך הצינסי הנקובים להיות להם שרש ברבוע הצינסי הבלתי נקובי' להיות להם שרש ככה יבא לשוות הדבר | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{x=\sqrt{\frac{48}{16}+\left(\frac{1}{4}\sdot\frac{72}{16^2}\right)}-\sqrt{\frac{1}{4}\sdot\frac{72}{16^2}}=\sqrt{3+\left(\frac{1}{4}\sdot\frac{72}{256}\right)}-\sqrt{\frac{1}{4}\sdot\frac{72}{256}}=\sqrt{3+\left(\frac{1}{4}\sdot\frac{9}{32}\right)}-\sqrt{\frac{1}{4}\sdot\frac{9}{32}}=\sqrt{3+\frac{9}{128}}-\sqrt{\frac{9}{128}}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :<math>\scriptstyle{\color{blue}{a=3x=3\sdot\sqrt{3+\frac{9}{128}}-\sqrt{\frac{9}{128}}=\sqrt{27+\frac{81}{128}}-\sqrt{\frac{81}{128}}}}</math> | ||
| + | |style="text-align:right;"|ואתה הנחת היות המספר ראשון ג' דברי‫'<br> | ||
| + | א"כ תכה ג' בשרש ג' וט' מקכ"ח פחות משרש ט' מקכ"ח ועולה שרש כ"ז ופ"א מקכ"ח פחות שרש מפ"א מקכ"ח וכן הוא המספר הראשון | ||
| + | |- | ||
| + | | | ||
| + | :<math>\scriptstyle{\color{blue}{b=4x=4\sdot\sqrt{3+\frac{9}{128}}-\sqrt{\frac{9}{128}}=\sqrt{49+{\color{red}{\frac{1}{8}}}}-\sqrt{1+\frac{1}{8}}}}</math> | ||
| + | |style="text-align:right;"|והמספר והשני הנחת היותו ד' דברים<br> | ||
| + | א"כ תכה ד' דברים בשרש ג' וט' מקכ"ח פחות שרש מט' מקכ"ח ועולה שרש ממ"ט פחות שרש מא' ושמינית וכן יבא להיות המספר השני | ||
| + | |- | ||
| + | |The equation can be restored to chapter 4: | ||
| + | |style="text-align:right;"|ודע שזאת ההשואה אפש' להשיבה אל הפרק הרביעי | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle16x^2+\sqrt{72}x=48</math> | ||
| + | |style="text-align:right;"|ויהיה לך י"ו צינסי וכך דברי' כמו שהוא שרש מע"ב שוים אל מ"ח | ||
| + | |- | ||
| + | |since <math>\scriptstyle\sqrt{x^2}=x</math> | ||
| + | |style="text-align:right;"|מפני כי שרש הצינסו יבא להיות הדבר | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== Chapter 40 ==== | ||
| + | |||
| + | |style="width:45%;text-align:right;"|<big>פרק מ‫'</big> | ||
| + | |- | ||
| + | |When cubes are equal to numbers and a root of numbers: | ||
| + | :<math>\scriptstyle ax^3=c+\sqrt{d}</math> | ||
| + | |style="text-align:right;"|עוד באופן אחר כאשר המעוקבים יהיו שוים אל מספרים ואל שרשי מספרים | ||
| + | |- | ||
| + | |The numbers should be divided by the number of the cubes. Keep the result. | ||
| + | |style="text-align:right;"|צריך לחלק המספר בכמות המעוק' ואשר יבא מזה שמרהו | ||
| + | |- | ||
| + | |Then, the number of the cubes should be multiplied by itself. | ||
| + | |style="text-align:right;"|אח"כ יוכו כמות המעוקבי' בעצמם | ||
| + | |- | ||
| + | |Divide the numbers that are the radicand by this product. | ||
| + | |style="text-align:right;"|ותחלק המספרי' הנקובי' להיות להם שרש באותה ההכאה | ||
| + | |- | ||
| + | |Add the root of the result to the quotient of the numbers that are not the radicand divided by the product of [the number of] the cubes. | ||
| + | |style="text-align:right;"|ושרש העולה מזה תחבר אל מה שעלה מחלוקת המספרי' הבלתי נקובי' היות להם שרש בכמות המעוקבי‫' | ||
| + | |- | ||
| + | |The cube root of this sum is equal to the thing. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{x=\sqrt[3]{\frac{c}{a}+\sqrt{\frac{d}{a^2}}}}}</math> | ||
| + | |style="text-align:right;"|ושרש מעו' מאותו הסך יבא לשוות הדברי‫' | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:a/b=⅔;(ab)b=100+\sqrt{8}|618|GBTu}}Find me two numbers such that the first is a part of the other as 2 is of 3; | ||
| + | :and when the first is multiplied by the second, then this product is multiplied by the second, it yields 100 and a root of 8. | ||
| + | :I ask: how much will each number be? | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle a:b=2:3\\\scriptstyle\left(a\sdot b\right)\sdot b=100+\sqrt{8}\end{cases}</math> | ||
| + | |style="text-align:right;"|עשה לי החשבון תמצא לי שני מספרים שיהיה המספר ראשון חלק מהאחר כמו שב' הוא מג‫'<br> | ||
| + | ובהכות הראשון בשני ואותה ההכאה תוכה בשני יעשה מאה ושרש ח‫'<br> | ||
| + | אשאל כמה יבא להיות כל אחד מהמספרי‫'{{#annotend:GBTu}} | ||
| + | |- | ||
| + | |The procedure: | ||
| + | |style="text-align:right;"|זהו מעשהו | ||
| + | |- | ||
| + | | | ||
| + | :defining: | ||
| + | :*the first number as two things <math>\scriptstyle{\color{blue}{a=2x}}</math> | ||
| + | |style="text-align:right;"|תניח שהמספר הראשון יהיה ב' דברים | ||
| + | |- | ||
| + | | | ||
| + | :*the other will be three things <math>\scriptstyle{\color{blue}{b=3x}}</math> | ||
| + | |style="text-align:right;"|והאחר יבא להיות ג' דברי‫' | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{\left(2x\sdot3x\right)\sdot3x=6x^2\sdot3x}}</math> | ||
| + | |style="text-align:right;"|עתה תכה הראשון בשני וזהו ב' דברי' בג' דברים ועולה ו' צינסי<br> | ||
| + | אח"כ תכה זאת ההכאה שהיא ו' צינסי במספר השני שהוא ג' דברי‫' | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle18x^3=100+\sqrt{8}</math> | ||
| + | |style="text-align:right;"|ועולה י"ח מעוקבי' והם שוים אל ק' ושרש ח‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Pursuing the above rule: | ||
| + | |style="text-align:right;"|עתה תרדוף כפי הכלל הנתון למעלה | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle x&\scriptstyle=\sqrt[3]{\frac{100}{18}+\sqrt{\frac{8}{18^2}}}\\&\scriptstyle=\sqrt[3]{\left(5+\frac{5}{9}\right)+\sqrt{\frac{8}{324}}}\\&\scriptstyle=\sqrt[3]{\left(5+\frac{5}{9}\right)+\sqrt{\frac{2}{81}}}\\\end{align}}}</math> | ||
| + | |style="text-align:right;"|תחלק המספר בכמות המעוקבי' וזהו ק' בי"ח ועולה מזה ה' וה' תשיעיות ושמרם | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|אח"כ תכה כמות המעוקבי' בעצמם והם י"ח שכ"ד | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ותחלק המספרי' הנקובי' שהם ח' בשכ"ד ועולה מזה ב' חלקים מפ"א | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ושרש ב' מפ"א תחבר אל מה שעלה בחלוקת המספרי' אשר לא נקבו להיות להם שרש בכמות המעוקבים שזהו על ה' וה' תשיעיות<br> | ||
| + | ועולה ה' וה' תשיעיות ושרש ב' מפ"א<br> | ||
| + | והשרש מעוקב מזה הסך יבא לשוות הדבר | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle a=2x&\scriptstyle=2\sdot\sqrt[3]{\left(5+\frac{5}{9}\right)+\sqrt{\frac{2}{81}}}\\&\scriptstyle=\sqrt[3]{8\sdot\left[\left(5+\frac{5}{9}\right)+\sqrt{\frac{2}{81}}\right]}\\&\scriptstyle=\sqrt[3]{\left(44+\frac{4}{9}\right)+\sqrt{1+\frac{47}{81}}}\\\end{align}}}</math> | ||
| + | |style="text-align:right;"|ואתה הנחת היות המספר ראשון ב' דברי‫'<br> | ||
| + | א"כ תכה ב' בשרש מעוקב מה' וה' תשיעיות מחובר עמם שרש ב' שמיניות | ||
| + | |- | ||
| + | | | ||
| + | :Remember that you must convert 2 into a cube root; you get a cube root of 8. | ||
| + | |style="text-align:right;"|ותזכור שהנך צריך להשיב ב' אל שרש מעוק' ויהיה לך שרש מעוק' מח‫' | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ותכה בשרש מעוק' מה' וה' תשיעיו‫'<br> | ||
| + | וזהו שרש ב' מפ"א עולה שרש מעוק' ממ"ד וד' תשיעיות<br> | ||
| + | תחבר עמו שרש א' ומ"ז מפ"א ושרש זה הסך יבא להיות המספר ראשון | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle b=3x&\scriptstyle=3\sdot\sqrt[3]{\left(5+\frac{5}{9}\right)+\sqrt{\frac{2}{81}}}\\&\scriptstyle=\sqrt[3]{\left[27\sdot\left(5+\frac{5}{9}\right)\right]+\sqrt{27^2\sdot\frac{2}{81}}}\\&\scriptstyle=\sqrt[3]{150+\sqrt{729\sdot\frac{2}{81}}}\\&\scriptstyle=\sqrt[3]{150+\sqrt{18}}\\\end{align}}}</math> | ||
| + | |style="text-align:right;"|והשני הנחת היותו ג' דברים<br> | ||
| + | א"כ תכה ג' בשרש מעוק' מה' וה' תשיעיו' מחובר עמם שרש ב' מפ"א | ||
| + | |- | ||
| + | | | ||
| + | :Remember that you must convert 3 into a cube root; you get a cube root of 27. | ||
| + | |style="text-align:right;"|ותזכור כי הנך צריך להשיב ג' אל שרש מעו' ויהיה לך שרש מעוק' מכ"ז | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|עתה תכה כ"ז בה' וה' תשיעיות ועולה ק"נ | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|עתה תכה כ"ז בעצמם ועולה תשכ"ט | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ותכה תשכ"ט בב' מפ"א עולה י"ח<br> | ||
| + | וזה הי"ח הוא שרש מי"ח<br> | ||
| + | ויהיה לך ק"נ ושרש מי"ח<br> | ||
| + | ושרש מעוק' מזה הסך שהוא מחובר ק"נ עם שרש י"ח יבא להיות המספר השני | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== Chapter 41 ==== | ||
| + | |||
| + | |style="width:45%;text-align:right;"|<big>פרק מ"א</big> | ||
| + | |- | ||
| + | |When numbers are equal to cubes and a root of cubes: | ||
| + | :<math>\scriptstyle c=ax^3+\sqrt{bx^3}</math> | ||
| + | |style="text-align:right;"|כאשר המספרי' יהיו שוים אל המעוקבי' ושרשי מעוקבי‫' | ||
| + | |- | ||
| + | |The numbers should be divided by the number of the cubes that are not the radicand. Keep the result. | ||
| + | |style="text-align:right;"|צריך לחלק המספרי' בכמות המעוקבי' אשר לא נקבו להיות להם שרש ומה שיבא מזה תשמור | ||
| + | |- | ||
| + | |Then, multiply [the number of] the cubes that are not the radicand by itself. | ||
| + | |style="text-align:right;"|אח"כ תכה המעו' אשר לא נקבו להיות להם שרש בעצמם | ||
| + | |- | ||
| + | |Divide the number of the cubes that are the radicand by this product. | ||
| + | |style="text-align:right;"|ותחלק כמות המעוק' הנקובי' להיות להם שרש באותה ההכאה | ||
| + | |- | ||
| + | |[Add] a quarter of the result to the quotient you kept. | ||
| + | |style="text-align:right;"|ורובע העולה מזה תחלק על החלוק אשר שמרת | ||
| + | |- | ||
| + | |The root of this sum minus the root of the quarter you added - meaning the root of a quarter of the quotient of the [number of] cubes that are the radicand divided by the product of the [number of] cubes that are not the radicand - is equal to the root of the cube. | ||
| + | |style="text-align:right;"|ושרש אותו הסך פחות שרש אותו הרובע אשר חברת רצו' שרש הא' רביע ממה שעלה בחלוק המעוק' {{#annot:term|1073,2561|Wjor}}שנקבו להיות להם שרש{{#annotend:Wjor}} בהכאת המעוק' אשר לא נקבו היות להם שרש יבא להיות | ||
| + | שרש המעוק‫' | ||
| + | |- | ||
| + | |Multiply this root by itself; the result is the cube. | ||
| + | |style="text-align:right;"|ותכה זה השרש בעצמו יבא להיות המעוקב | ||
| + | |- | ||
| + | |The cube root of this product is the thing. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{x=\sqrt[3]{\left(\sqrt{\frac{c}{a}+\left(\frac{1}{4}\sdot\frac{b}{a^2}\right)}-\sqrt{\frac{1}{4}\sdot\frac{b}{a^2}}\right)^2}}}</math> | ||
| + | |style="text-align:right;"|ושרש המעו' מזאת ההכאה יבא להיות הדבר | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:a/b=⅔;a²b+\sqrt{a²b}=342|618|oR0N}}Find me two numbers such that the first is a part of the second as 2 is of 3; | ||
| + | :and if the first is multiplied by itself, then this product is multiplied by the second, and this product is summed with its root, it yields 342. | ||
| + | :I ask: how much will each number be? | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle a:b=2:3\\\scriptstyle\left(a^2\sdot b\right)+\sqrt{a^2\sdot b}=342\end{cases}</math> | ||
| + | |style="text-align:right;"|עשה לי זה החשבון תמצא לי שני מספרי' שיהיה הראשון חלק מהשני כמו שב' הוא מג‫'<br> | ||
| + | ומוכה הראשון בעצמו ואותה ההכאה תכה בשני ותחובר זאת ההכאה עם שרשה יעשה שמ"ב<br> | ||
| + | אשאל כמה יבא להיות כל אחד מהמספרי‫'{{#annotend:oR0N}} | ||
| + | |- | ||
| + | |The procedure: | ||
| + | |style="text-align:right;"|זהו מעשהו | ||
| + | |- | ||
| + | | | ||
| + | :defining: | ||
| + | :*the first number as two things <math>\scriptstyle{\color{blue}{a=2x}}</math> | ||
| + | |style="text-align:right;"|תניח שהמספר ראשון יהיה ב' דברי‫' | ||
| + | |- | ||
| + | | | ||
| + | :*the other must be three things <math>\scriptstyle{\color{blue}{b=3x}}</math> | ||
| + | |style="text-align:right;"|והאחר מחוייב שיהיה ג' דברי‫' | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{\left(2x\right)^2\sdot3x=4x^2\sdot3x}}</math> | ||
| + | |style="text-align:right;"|עתה תכה המספר הראשון שהוא ב' דברי' בעצמו ועולה ד' צינסי<br> | ||
| + | ואלו ד' צינסי תכם על המספר השני שזהו על ג' דברי‫' | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle12x^3+\sqrt{12x^3}=342</math> | ||
| + | |style="text-align:right;"|ועולה י"ב מעוקבי' ושרש י"ב מעוק' יבאו להיות שוים אל שמ"ב | ||
| + | |- | ||
| + | | | ||
| + | ::Pursuing the above rule: | ||
| + | |style="text-align:right;"|עתה תרדוף כפי הכלל הנתון למעלה | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle x&\scriptstyle=\sqrt[3]{\left(\sqrt{\frac{342}{12}+\left(\frac{1}{4}\sdot\frac{12}{12^2}\right)}-\sqrt{\frac{1}{4}\sdot\frac{12}{12^2}}\right)^2}\\&\scriptstyle=\sqrt[3]{\left(\sqrt{\left(28+\frac{1}{2}\right)+\left(\frac{1}{4}\sdot\frac{12}{144}\right)}-\sqrt{\frac{1}{4}\sdot\frac{12}{144}}\right)^2}\\&\scriptstyle=\sqrt[3]{\left(\sqrt{\left(28+\frac{1}{2}\right)+\left(\frac{1}{4}\sdot\frac{1}{12}\right)}-\sqrt{\frac{1}{4}\sdot\frac{1}{12}}\right)^2}\\&\scriptstyle=\sqrt[3]{\left(\sqrt{\left(28+\frac{1}{2}\right)+\frac{1}{48}}-\sqrt{\frac{1}{48}}\right)^2}\\&\scriptstyle=\sqrt[3]{\left(\sqrt{28+\frac{25}{48}}-\sqrt{\frac{1}{48}}\right)^2}=\sqrt[3]{27}=3\\\end{align}}}</math> | ||
| + | |style="text-align:right;"|תחלק המספרי' בכמות המעוק' שזהו שמ"ב על י"ב ויבא מזה כ"ח וחצי ושמרם | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|אח"כ תכה כמות המעוק' אשר לא נקבו להיות להם שרש והם י"ב בעצמם ועולה קמ"ד | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ותחלק כמות המעוקבי' הנקובי' להיות להם שרש שהם הי"ב האחד בקמ"ד ויעלה מזה א' מי"ב | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ותקח הרביע מזה הא' חלק מי"ב שהוא א' חלק ממ"ח | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ותחברהו עם כ"ח וחצי אשר שמרת ויהיה לך כ"ח וכ"ה ממ"ח | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ושרש כ"ח וכ"ה ממ"ח פחות שרש אותו הרביע דהיינו פחות שרש א' ממ"ח יבא להיות שרש המעוקב | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ותכה זה השרש בעצמו רצוני שרש כ"ח וכ"ה ממ"ח פחות שרש א' ממ"ח בעצמו<br> | ||
| + | ועולה כ"ז וככה יבא להיות המעוק‫'<br> | ||
| + | ושרשו המעוק' שהוא ג' יבא להיות הדבר | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{a=2x=2\sdot3=6}}</math> | ||
| + | |style="text-align:right;"|ואתה הנחת היות המספר ראשון ב' דברי‫'<br> | ||
| + | א"כ תכה ב' במה ששוה הדבר וזהו ג' ועולה ו' וכן יבא להיות המספר ראשון | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{b=3x=3\sdot3=9}}</math> | ||
| + | |style="text-align:right;"|והשני הנחת היותו ג' דברי‫'<br> | ||
| + | א"כ תכה ג' בג' ועולה ט' וככה יבא להיות המספר השני | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== Chapter 42 ==== | ||
| + | |||
| + | |style="width:45%;text-align:right;"|<big>פרק מ"ב</big> | ||
| + | |- | ||
| + | |When squares of squares are equal to a number and a root of a number: | ||
| + | :<math>\scriptstyle ax^4=c+\sqrt{d}</math> | ||
| + | |style="text-align:right;"|עוד כאשר הצינסי מצינסי יהיו שוים אל מספר ושרש מספר | ||
| + | |- | ||
| + | |The numbers should be divided by the number of the squares of squares. Keep the result. | ||
| + | |style="text-align:right;"|צריך לחלק המספרי' בכמות הצינסי מצינסי ומה שיעלה ישמור | ||
| + | |- | ||
| + | |Then, the number of the squares of squares should be multiplied by itself. | ||
| + | |style="text-align:right;"|ואח"כ יוכה כמות צינסי מצינסי בעצמו | ||
| + | |- | ||
| + | |The numbers that are the radicand should be divided by this product. | ||
| + | |style="text-align:right;"|ולחלק המספרי' הנקובי' להיות להם שרש באותה ההכאה | ||
| + | |- | ||
| + | |The root of the result is added to the quotient we kept, which is the result of division of the numbers that are not the radicand by the number of the squares of squares. | ||
| + | |style="text-align:right;"|ושרש מה שיבא מזה יחובר אל החלוק אשר שמרנו וזהו מה שבא בחלוק המספרי' אשר לא נקבו היות להם שרש בכמות הצינסי מצינסי | ||
| + | |- | ||
| + | |The root of the root of this sum is equal to the thing. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{x=\sqrt[4]{\frac{c}{a}+\sqrt{\frac{d}{a^2}}}}}</math> | ||
| + | |style="text-align:right;"|ושרש השרש מאותו הסך יבא לשוות הדבר | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:a/b=⅔;(ab)²=20+√30|618|9iel}}[Find me two numbers] such that one is a part of the other as 2 is of 3; | ||
| + | :and when the first is multiplied by the second and this product is multiplied by itself, it yields 20 and a root of 20. | ||
| + | :I ask: how much will each number be? | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle a:b=2:3\\\scriptstyle\left(a\sdot b\right)^2=20+\sqrt{30}\end{cases}</math> | ||
| + | |style="text-align:right;"|עשה לי זה החשבון שיהיה האחד חלק מהאחר כמו שב' הוא חלק מג‫'<br> | ||
| + | ובהכות הראשון בשני ואותה ההכאה תוכה בעצמה יעשה כ' ושרש ל‫'<br> | ||
| + | אשאל כמה יבא להיות כל אחד מהמספרי‫'{{#annotend:9iel}} | ||
| + | |- | ||
| + | |The procedure: | ||
| + | |style="text-align:right;"|זהו מעשהו | ||
| + | |- | ||
| + | | | ||
| + | :Suppose the first number is two things [<math>\scriptstyle{\color{blue}{a=2x}}</math>] and the second is three things [<math>\scriptstyle{\color{blue}{b=3x}}</math>]. | ||
| + | |style="text-align:right;"|תניח שהמספר ראשון יהיה ב' דברי' והשני ג' דברים | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{\left(2x\sdot3x\right)^2=\left(6x^2\right)^2}}</math> | ||
| + | |style="text-align:right;"|עתה תכה הראשון בשני וזהו ב' בג' ועולה ו' צינסי<br> | ||
| + | ואלו הו' צינסי תכם בעצמם | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle36x^4=20+\sqrt{30}</math> | ||
| + | |style="text-align:right;"|ויעלו ל"ו צינסי מצינסי אשר הם שוים אל כ' ושרש ל‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Pursuing the above rule: | ||
| + | |style="text-align:right;"|עתה תרדוף כפי הכלל האמור למעלה | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle x&\scriptstyle=\sqrt[4]{\frac{20}{36}+\sqrt{\frac{30}{36^2}}}\\&\scriptstyle=\sqrt[4]{\frac{5}{9}+\sqrt{\frac{30}{1296}}}\\&\scriptstyle=\sqrt[4]{\frac{5}{9}+\sqrt{\frac{5}{216}}}\\\end{align}}}</math> | ||
| + | |style="text-align:right;"|תחלק המספרי' על כמות הצינסי מצינסי שזהו כ' על ל"ו שבא מזה ה' תשיעיות ושמרם | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|אח"כ תכה כמות הצינסי מצינסי בעצמם וזהו ל"ו ועולה אלף רצ"ו | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ותחלק המספרי' הנקובי' להיות להם שרש שהם ל' על אלף ורצ"ו ועולה מזה ה' מרי"ו | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ושרש אלו הרי"ו תחבר אל החלוק אשר שמרת שהוא על ה' תשיעיות<br> | ||
| + | ויהיה לך ה' תשיעיות ושרש ה' מרי"ו<br> | ||
| + | ושרש השרש מזה הסך יבא לשוות הדבר | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle a=2x&\scriptstyle=2\sdot\sqrt[4]{\frac{5}{9}+\sqrt{\frac{5}{216}}}\\&\scriptstyle=\sqrt[4]{\left(8+\frac{8}{9}\right)+\sqrt{5+\frac{25}{27}}}\\\end{align}}}</math> | ||
| + | |style="text-align:right;"|ואתה הנחת היות המספר ראשון ב' דברים<br> | ||
| + | א"כ תכה ב' בשרש שרש ה' מט' מחובר עם שרש ה' מרי"ו<br> | ||
| + | ועולה שרש שרש ח' וח' מט' מחובר עם שרש ה' וכ"ה מכ"ז וככה יבא להיות המספר ראשון | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle b=3x&\scriptstyle=3\sdot\sqrt[4]{\frac{5}{9}+\sqrt{\frac{5}{216}}}\\&\scriptstyle=\sqrt[4]{45+\sqrt{151+\frac{189}{216}}}\\\end{align}}}</math> | ||
| + | |style="text-align:right;"|והשני הנחת היותו ג' דברי‫'<br> | ||
| + | א"כ תכה ג' בשרש שרש ה' מט' מחובר עם שרש ה' מרי"ו<br> | ||
| + | ועולה שרש משרש מ"ה מחובר עם שרש קנ"א וקפ"ט מרי"ו וככה יבא להיות המספר השני | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== Chapter 43 ==== | ||
| + | |||
| + | |style="width:45%;text-align:right;"|<big>פרק מ"ג</big> | ||
| + | |- | ||
| + | |{{#annot:c=ax⁴+√(bx⁴)|719|h9il}}When numbers are equal to squares of squares and a root of squares of squares: | ||
| + | :<math>\scriptstyle c=ax^4+\sqrt{bx^4}</math> | ||
| + | |style="text-align:right;"|כאשר המספרי' יהיו שוים אל הצינסי מצינסי ואל שרשי צינסי מצינסי{{#annotend:h9il}} | ||
| + | |- | ||
| + | |The numbers should be divided by the number of the squares of squares that are not the radicand. Keep the result. | ||
| + | |style="text-align:right;"|צריך לחלק המספרי' על כמות הצינסי מצינסי אשר לא נקבו להיות להם שרש ומה שיבא מזה תשמור | ||
| + | |- | ||
| + | |Then, multiply the number of the squares of squares that are not the radicand by itself. | ||
| + | |style="text-align:right;"|ואח"כ תכה כמות הצינסי מצינסי אשר לא נקבו להיות להם שרש בעצמם | ||
| + | |- | ||
| + | |The [number of the] squares of squares that are the radicand should be divided by this product. | ||
| + | |style="text-align:right;"|ולחלוק הצינסי מצינסי הנקובים להיות להם שרש באותה ההכאה | ||
| + | |- | ||
| + | |Add a quarter of the result to the quotient of the numbers divided by the number of the squares of squares. | ||
| + | |style="text-align:right;"|ורביע ממה שיבא תחבר אל החלוק שבא לך מהמספרים על כמות הצינסי מצינסי | ||
| + | |- | ||
| + | |The root of this sum minus the root of the quarter is equal to the square. | ||
| + | |style="text-align:right;"|ושרש ממרובע מהסך ההוא פחות שרש אותו הרביע אשר יבא לשוות הצינסו | ||
| + | |- | ||
| + | |The root of this remainder is equal to the thing; that is the root of the quarter subtracted from the root of the sum and the root of the remainder is equal to the thing. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{x=\sqrt{\sqrt{\frac{c}{a}+\left(\frac{1}{4}\sdot\frac{b}{a^2}\right)}-\sqrt{\frac{1}{4}\sdot\frac{b}{a^2}}}}}</math> | ||
| + | |style="text-align:right;"|ושרש זה השארית יבא לשוות הדבר<br> | ||
| + | וזהו שרש אותו הרביע {{#annot:term|181,2562|zCID}}מוצא מ{{#annotend:zCID}}שרש הסך שרש הנשאר יבא להיות שוה הדבר | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:a/b=⅔;(ab)²+a²√4=4¼|618|jPKB}}Find me two numbers such that one is a part of the other as 2 is of 3; | ||
| + | :and when the first is multiplied by the second and this product is multiplied by itself then summed with the product of the first multiplied by itself then by the root of 4, it yields 4 and a quarter. | ||
| + | :I ask: how much will each number be? | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle a:b=2:3\\\scriptstyle\left(a\sdot b\right)^2+\left(a^2\sdot\sqrt{4}\right)=4+\frac{1}{4}\end{cases}</math> | ||
| + | |style="text-align:right;"|עשה לי זה החשבון תמצא לי שני מספרי' שיהיה הא' חלק מהאחר כמו שב' הוא מג‫'<br> | ||
| + | ומוכה הראשון בשני וזאת ההכאה תוכה בעצמה ותחובר זאת ההכאה עם הכאת הראשון מוכה בעצמו ומה שיבא בשרש ד' יעשה ד' ורביע<br> | ||
| + | אשאל כמה יבא להיות כל אחד מהמספרי‫'{{#annotend:jPKB}} | ||
| + | |- | ||
| + | |The procedure: | ||
| + | |style="text-align:right;"|זהו מעשהו | ||
| + | |- | ||
| + | | | ||
| + | :Suppose the first number is two things [<math>\scriptstyle{\color{blue}{a=2x}}</math>] and the second is three things [<math>\scriptstyle{\color{blue}{b=3x}}</math>]. | ||
| + | |style="text-align:right;"|תניח שהמספר הראשון יהיה ב' דברי' והשני יהיה ג' דברים | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{\left(2x\sdot3x\right)^2=\left(6x^2\right)^2=36x^4}}</math> | ||
| + | |style="text-align:right;"|עתה תכה הראשון בשני וזהו ב' דברים בג' דברי' ועולה ו' צינסי<br> | ||
| + | וזאת ההכאה שהוא ו' צינסי תכה בעצמה ועולה ל"ו צינסי מצינסי ותשמרם | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{\left(2x\right)^2\sdot\sqrt{4}=4x^2\sdot\sqrt{4}=\sqrt{64x^4}}}</math> | ||
| + | |style="text-align:right;"|אח"כ תכה המספר ראשון שהוא ב' דברים בעצמו ועולה ד' צינסי<br> | ||
| + | ואלו הד' צינסי תכה בשרש ד' ועולה שרש מס"ד צינסי מצינסי | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{\sqrt{64x^4}+36x^4}}</math> | ||
| + | |style="text-align:right;"|עתה תחבר שרש מס"ד צינסי מצינסי עם מה ששמרת שזהו עם ל"ו צינסי מצינסי | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle36x^4+\sqrt{64x^4}=4+\frac{1}{4}</math> | ||
| + | |style="text-align:right;"|ויהיה לך ל"ו צינסי מצינסי ושרש מס"ד צינסי מצינסי היות שוים אל ד' ורביע | ||
| + | |- | ||
| + | | | ||
| + | ::Pursuing the above rule: | ||
| + | |style="text-align:right;"|עתה תרדוף כפי הכלל האמור למעלה | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle x&\scriptstyle=\sqrt{\sqrt{\frac{4+\frac{1}{4}}{36}+\left(\frac{1}{4}\sdot\frac{64}{36^2}\right)}-\sqrt{\frac{1}{4}\sdot\frac{64}{36^2}}}\\&\scriptstyle=\sqrt{\sqrt{\frac{17}{144}+\left(\frac{1}{4}\sdot\frac{64}{1296}\right)}-\sqrt{\frac{1}{4}\sdot\frac{64}{1296}}}\\&\scriptstyle=\sqrt{\sqrt{\frac{17}{144}+\left(\frac{1}{4}\sdot\frac{4}{81}\right)}-\sqrt{\frac{1}{4}\sdot\frac{4}{81}}}\\&\scriptstyle=\sqrt{\sqrt{\frac{17}{144}+\frac{1}{81}}-\sqrt{\frac{1}{81}}}\\&\scriptstyle=\sqrt{\sqrt{\frac{169}{1296}}-\sqrt{\frac{1}{81}}}\\\end{align}}}</math> | ||
| + | |style="text-align:right;"|תחלק המספרים על כמות הצינסי מצינסי אשר לא נקבו היות להם שרש שזהו ד' ורביע בל"ו ויבא מזה י"ז מקמ"ד ותשמרם | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|אח"כ תכה כמות הצינסי מצינסי אשר לא נקבו היות להם שרש בעצמם וזהו ל"ו ועולה אלף ורצ"ו | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ותחלק הצינסי מצינסי הנקובי' להיות להם שרש שזהו ס"ד באלף ורצ"ו ויבא מזה ד' מפ"א | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|עתה תקח הרביע מאלו הד' מפ"א ויהיה לך א' מפ"א | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|תחברהו על י"ז מקמ"ד שבאו בחלוקת המספרים על כמות הצינסי מצינסי וזהו אשר שמרת ויהיה לך קס"ט מאלף ורצ"ו | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ושרש מקס"ט מאלף ורצ"ו פחות שרש מזה הא' מפ"א<br> | ||
| + | שזהו הרביע ממה שבא לך בחלקך הצינסי מהצינסי הנקובים להיות להם שרש על הכאת הצינסי מצינסי אשר לא נקבו להיות להם שרש<br> | ||
| + | יבא להיות שוה הצינסו<br> | ||
| + | ושרש זה השארית יבא להיות הדבר | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle a=2x&\scriptstyle=2\sdot\sqrt{\sqrt{\frac{169}{1296}}-\sqrt{\frac{1}{81}}}\\&\scriptstyle=\sqrt{\sqrt{16\sdot\frac{169}{1296}}-\sqrt{16\sdot\frac{1}{81}}}\\&\scriptstyle=\sqrt{\sqrt{\frac{2704}{1296}}-\sqrt{\frac{16}{81}}}\\&\scriptstyle=\sqrt{\sqrt{2+\frac{7}{81}}-\sqrt{\frac{16}{81}}}\\&\scriptstyle=\sqrt{\left(1+\frac{4}{9}\right)-\frac{4}{9}}\\&\scriptstyle=\sqrt{1}=1\\\end{align}}}</math> | ||
| + | |style="text-align:right;"|ואתה הנחת שהמספר ראשון היה ב' דברים<br> | ||
| + | א"כ תכה ב' בשרש השארית הנשאר מהוצאת שרש א' מפ"א חוץ משרש קס"ט מאלף רצ"ו | ||
| + | |- | ||
| + | | | ||
| + | :Remember that you must convert 2 into a root of a root; you get a root of a root of 16. | ||
| + | |style="text-align:right;"|ותזכור כי הנך צריך להשיב ב' אל שרש משרש ויהיה לך שרש משרש י"ו | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ותכה אלו הי"ו בקס"ט מאלף ורצ"ו ועולה אלפיים ותש"ד מאלף ורצ"ו<br> | ||
| + | שהם ב' וז' מפ"א ושרש מזה תשמור | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ואח"כ תכה י"ו בא' מפ"א ועולה י"ו מפ"א | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ושרש זה הי"ו מפ"א תוציא מהשרש אשר שמרת שהוא מב' וז' מפ"א | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ושרש הנשאר יבא להיות המספר ראשון | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ואם רצית להשיבו אל שלמים<br> | ||
| + | דע כי שרש ב' וז' תשיעיות הוא א' וד' מט‫'<br> | ||
| + | ושרש מי"ו מפ"א יבא להיות ד' תשיעיות<br> | ||
| + | ולהוציא ד' מט' מא' וד' מט' ישאר אחד<br> | ||
| + | ושרש זה הנשאר והוא א' יבא להיות המספר הראשון | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle b=3x&\scriptstyle=3\sdot\sqrt{\sqrt{\frac{169}{1296}}-\sqrt{\frac{1}{81}}}\\&\scriptstyle=\sqrt{\sqrt{10+\frac{9}{16}}-\sqrt{1}}\\&\scriptstyle=\sqrt{\left(3+\frac{1}{4}\right)-1}\\&\scriptstyle=\sqrt{2+\frac{1}{4}}=1+\frac{1}{2}\\\end{align}}}</math> | ||
| + | |style="text-align:right;"|והשני הנחת היותו ג' דברי‫'<br> | ||
| + | א"כ תכה ג' במה ששוה הדבר<br> | ||
| + | ויהיה לך שרש ממוצא שרש א' חוץ משרש עשרה וט' מי"ו וככה יהיה המספר השני<br> | ||
| + | א"כ תוציא שרש א' שהוא א' חוץ מג' ורביע שהוא שרש מי' וט' מי"ו<br> | ||
| + | ישאר ב' וא' מד‫'<br> | ||
| + | ושרש זה השארית שהוא א' וחצי יבא להיות המספר השני | ||
| + | |- | ||
| + | |This calculation can be restored to another chapter | ||
| + | |style="text-align:right;"|ודע כי זה החשבון היה יכול לבוא אל פרק אחר | ||
| + | |- | ||
| + | | | ||
| + | ::multiplying the square by 2 which is the root of 4: <math>\scriptstyle{\color{blue}{a^2\sdot\sqrt{4}=a^2\sdot2}}</math> | ||
| + | |style="text-align:right;"|אם היית מכה הכאת המספר הראשון שהיתה ד' צינסי בב' שהוא שרש ד‫' | ||
| + | |- | ||
| + | | | ||
| + | ::in the present chapter the square was multiplied by the root of 4, as if there was no expressible root for 4 | ||
| + | |style="text-align:right;"|אבל ברצותנו להשיבו אל זה הפרק הוכה בשרש ד' כאלו לא היה אל ד' שרש מדבר | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== Chapter 44 ==== | ||
| + | |||
| + | |style="width:45%;text-align:right;"|<big>פרק מ"ד</big> | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|עוד באופן אחר | ||
| + | |- | ||
| + | |{{#annot:ax⁴+bx²=c|719|Tu7N}}When squares of squares plus squares are equal to a number: | ||
| + | :<math>\scriptstyle ax^4+bx^2=c</math> | ||
| + | |style="text-align:right;"|כאשר הצינסי מצינסי וצינסי יהיו שוים אל מספר{{#annotend:Tu7N}} | ||
| + | |- | ||
| + | |The whole equation should be divided by the number of the squares of the squares. | ||
| + | |style="text-align:right;"|צריך לחלק כל ההשואה על כמות הצינסי מצינסי | ||
| + | |- | ||
| + | |Then, the number of the squares should be halved. | ||
| + | |style="text-align:right;"|ואח"כ לחלק כמות הצינסי לחצי | ||
| + | |- | ||
| + | |[One] half should be multiplied by itself. | ||
| + | |style="text-align:right;"|ולהכות כל אחד מהחצאים בעצמו | ||
| + | |- | ||
| + | |Add the product to the number. | ||
| + | |style="text-align:right;"|והעולה מזה תחבר על המספר | ||
| + | |- | ||
| + | |Subtract the other half of the number of the squares from the root of the sum. | ||
| + | |style="text-align:right;"|ומשרש הסך תוציא המחצית האחר מכמות הצינסי | ||
| + | |- | ||
| + | |The root of the remainder is equal to the thing. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{x=\sqrt{\sqrt{\left(\frac{1}{2}\sdot\frac{b}{a}\right)^2+\frac{c}{a}}-\left(\frac{1}{2}\sdot\frac{b}{a}\right)}}}</math> | ||
| + | |style="text-align:right;"|ושרש הנשאר יבא לשוות הדבר | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:a²=b-5;a²+b²=100|618|a55J}}Find me two numbers, such that when the first is multiplied by itself it yields the second minus 5, and when each of them is multiplied by itself, then both products are summed together, it yields 100. | ||
| + | :I ask: how much will each of the numbers be? | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle a^2=b-5\\\scriptstyle a^2+b^2=100\end{cases}</math> | ||
| + | |style="text-align:right;"|ואשים לך המשל ואומ' כן עשה לי זה החשבון תמצא לי שני מספרי' שכאשר יוכה הראשון בעצמו יעשה השני פחות ה‫'<br> | ||
| + | ויוכו כל אחד מהם בעצמו ויחוברו אותם ההכאות יחד יעשה ק‫'<br> | ||
| + | ואשאל יבא כמה להיות כל אחד מהמספרים{{#annotend:a55J}} | ||
| + | |- | ||
| + | | | ||
| + | :This is its procedure: | ||
| + | |style="text-align:right;"|זהו מעשהו | ||
| + | |- | ||
| + | | | ||
| + | :Suppose the first number is a thing [<math>\scriptstyle{\color{blue}{a=x}}</math>]. | ||
| + | |style="text-align:right;"|תניח שהמספר הראשון יהיה דבר אחד | ||
| + | |- | ||
| + | | | ||
| + | :Multiply it by itself; it is 1 square. | ||
| + | :<math>\scriptstyle{\color{blue}{a^2=x^2}}</math> | ||
| + | |style="text-align:right;"|ותכהו בעצמו והיה א' צינסו | ||
| + | |- | ||
| + | | | ||
| + | :Then, the second is 1 square plus 5 numbers. | ||
| + | :<math>\scriptstyle{\color{blue}{b=x^2+5}}</math> | ||
| + | |style="text-align:right;"|א"כ יבא להיות השני א' צינסו וה' מספרים | ||
| + | |- | ||
| + | | | ||
| + | :Multiply each of them by itself: | ||
| + | |style="text-align:right;"|עתה תכה כל אחד מהם בעצמו | ||
| + | |- | ||
| + | | | ||
| + | :The first, which is one thing, becomes 1 square. | ||
| + | :<math>\scriptstyle{\color{blue}{x\sdot x=x^2}}</math> | ||
| + | |style="text-align:right;"|ועולה הראשון שהוא דבר אחד א' צינסו | ||
| + | |- | ||
| + | | | ||
| + | :The product of second, which is 1 square plus 5 numbers, by itself is 1 square of a square, 10 squares, and 25 numbers. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(x^2+5\right)^2=x^4+10x^2+25}}</math> | ||
| + | |style="text-align:right;"|והשני שהוא א' צינסו וה' מספרים עולה הכאתו בעצמו א' צינסו מצינסו וי' צינסי וכ"ה מספרי‫' | ||
| + | |- | ||
| + | | | ||
| + | :Sum up both products together; you get 1 square of a square, 11 squares, and 25 numbers that are equal to 100. | ||
| + | :<math>\scriptstyle{\color{blue}{x^4+11x^2+25=100}}</math> | ||
| + | |style="text-align:right;"|ותחבר שתי אלו ההכאות יחד ויהיה לך א' צינסו מצינסו וי"א צינסי וכ"ה מספרים אשר יבאו להיות שוים אל ק‫' | ||
| + | |- | ||
| + | | | ||
| + | :Now, subtract the number that is smaller in one of the sides [of the equation] from both sides [of the equation]. | ||
| + | :<math>\scriptstyle{\color{blue}{x^4+11x^2+25-25=100-25}}</math> | ||
| + | |style="text-align:right;"|עתה הוצא המספרים שהם פוחתים בכמות מאחד מהחלקים מכל אחד מהחלקים | ||
| + | |- | ||
| + | | | ||
| + | :One side remains 1 square of a square plus 11 squares without a number, which is equal to the other side that remains 75 numbers. | ||
| + | :<math>\scriptstyle{\color{blue}{x^4+11x^2=75}}</math> | ||
| + | |style="text-align:right;"|וישאר אחד מהחלקים א' צינסו מצינסו וי"א צינסי בלתי מספר שוה לחלק האחר אשר ישאר ע"ה מספרים | ||
| + | |- | ||
| + | | | ||
| + | :Pursue the above mentioned rule: | ||
| + | |style="text-align:right;"|עתה תרדוף כפי הכלל האמור למעלה | ||
| + | |- | ||
| + | | | ||
| + | :<span style=color:Green>'''Normalization''':</span> divide the whole equation by the number of the squares of the squares, which is one; the result is the equation itself. | ||
| + | :<math>\scriptstyle{\color{blue}{x^4+11x^2=75\quad/\div1}}</math> | ||
| + | |style="text-align:right;"|תחלק כל ההשואה על כמות הצינסי מצינסי שהוא אחד ויבא מזה ההשואה ההיא בעצמה | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|אח"כ תחלק כמות הצינסי לחצי וזהו י"א ויבא מזה ה' וחצי | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ואכה אלו הה' וחצי בעצמם ועולה ל' ורביע | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|וחברם על המספרים שזהו על ע"ה ויהיה לך ק"ה ורביע | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ומשרש אלו הק"ה ורביע הוצא מחצית הצינסי שהוא ה' וחצי וישאר שרש ק"ה ורביע פחות ה' וחצי | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ושרש זה הנשאר יבא להיות הדבר וזהו המספר הראשון | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle a=x&\scriptstyle=\sqrt{\sqrt{\left(\frac{11}{2}\right)^2+75}-\frac{11}{2}}=\sqrt{\sqrt{\left(5+\frac{1}{2}\right)^2+75}-\left(5+\frac{1}{2}\right)}=\sqrt{\sqrt{\left(30+\frac{1}{4}\right)+75}-\left(5+\frac{1}{2}\right)}\\&\scriptstyle=\sqrt{\sqrt{105+\frac{1}{4}}-\left(5+\frac{1}{2}\right)}\\\end{align}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|עתה תכה שרש זה השארית בעצמו וזהו שרש מה' וחצי משרש ק"ה ורביע בשרש מה' וחצי משרש ק"ה ורביע<br> | ||
| + | ועולה שרש מק"ה ורביע פחות ה' וחצי<br> | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ועתה אמ' קודם בשאלה שהמספר ראשון מוכה בעצמו ראוי שיעשה השני פחות ה‫'<br> | ||
| + | א"כ יבא לעשות השני ה' יותר מהכאת הראשון<br> | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ולכן תוסיף על הכאת הראשון שזהו על שרש ק"ה ורביע פחות ה' וחצי האמור<br> | ||
| + | ויהיה לך שרש ק"ה ורביע פחות חצי וכן יבא להיות המספר השני | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle b=x^2+5&\scriptstyle=\left[\sqrt{\sqrt{105+\frac{1}{4}}-\left(5+\frac{1}{2}\right)}\right]^2+5=\sqrt{105+\frac{1}{4}}-\left(5+\frac{1}{2}\right)+5\\&\scriptstyle=\sqrt{105+\frac{1}{4}}-\frac{1}{2}\\\end{align}}}</math> | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== Chapter 45 ==== | ||
| + | |- | ||
| + | | | ||
| + | ===== Chapter 45-I ===== | ||
| + | |||
| + | |style="width:45%;text-align:right;"|<big>פרק מ"ה</big> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|עוד אחר באופן אחר | ||
| + | |- | ||
| + | |{{#annot:bx²=ax⁴+c|719|tkSO}}When squares are equal to squares of squares and a root of a number: | ||
| + | :<math>\scriptstyle bx^2=ax^4+c</math> | ||
| + | |style="text-align:right;"|כאשר הצינסי יהיו שוים אל הצינסי מצינסי ואל מספר{{#annotend:tkSO}} | ||
| + | |- | ||
| + | |The whole equation should be divided by the number of the squares of squares. | ||
| + | |style="text-align:right;"|צריך לחלק כל ההשואה על כמות הצינסי מצינסי | ||
| + | |- | ||
| + | |Then, the number of the squares should be halved. | ||
| + | |style="text-align:right;"|ואח"כ לחלק כמות הצינסי לחצי | ||
| + | |- | ||
| + | |One half should be multiplied by itself. | ||
| + | |style="text-align:right;"|ולהכות אחד מהחצאים על עצמו | ||
| + | |- | ||
| + | |Subtract the number from the product. | ||
| + | |style="text-align:right;"|ומאותה ההכאה תוציא המספרים | ||
| + | |- | ||
| + | |Add the root of the remainder to the other half of [the number of] the squares. | ||
| + | |style="text-align:right;"|ושרש הנשאר תחבר על המחצית האחר מהצינסי | ||
| + | |- | ||
| + | |The root of the sum is the thing. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{x=\sqrt{\left(\frac{1}{2}\sdot\frac{b}{a}\right)+\sqrt{\left(\frac{1}{2}\sdot\frac{b}{a}\right)^2-\frac{c}{a}}}}}</math> | ||
| + | |style="text-align:right;"|ושרש אותו הסך יבא להיות הדבר | ||
| + | |- | ||
| + | |<math>\scriptstyle x=\sqrt{\left(\frac{1}{2}\sdot\frac{b}{a}\right)-\sqrt{\left(\frac{1}{2}\sdot\frac{b}{a}\right)^2-\frac{c}{a}}}</math> | ||
| + | |style="text-align:right;"|וחשבונות מה צריכי' לענות שהדבר ישוה שרש הנשאר בהוציא המספרי' מהכאת מחצית כמות הצינסי<br> | ||
| + | ושרש הנשאר ההוא יוציא מהחצי האחר מהצינסי | ||
| + | |- | ||
| + | |In the first answer the root of the remainder is added to half the squares, and the root of the sum is the result. | ||
| + | |style="text-align:right;"|וזהו כמו שבתשובה הראשונה יחובר שרש הנשאר על החצי האחר מהצינסי<br> | ||
| + | ושרש הסך ההוא יבא להיות הדבר | ||
| + | |- | ||
| + | |Likewise, the root of the remainder is subtracted from half the squares, and the root of this remainder is the result. | ||
| + | |style="text-align:right;"|כמו כן יוצא להפך שרש מאותו שנשאר ממחצית כמות הצינסי<br> | ||
| + | ושרש אותו השארית יבא לשוות הדבר | ||
| + | |- | ||
| + | |Many calculations can be solved by these procedures | ||
| + | |style="text-align:right;"|וחשבונות רבים אפש' לענות בם היות הדבר כמו שכל אחד מהאופנים אומר | ||
| + | |- | ||
| + | |Example: | ||
| + | |style="text-align:right;"|והנני {{#annot:term|898,1896|eUje}}אשים לך משל לפניך{{#annotend:eUje}} כמו שתוכל לראות לפנים | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:a/b=¾;(ab)²+27=9b²|618|gmur}}Find me two numbers such that the first is a part of the other as 3 is of 4; | ||
| + | :and the first multiplied by the second, and this product is multiplied by itself and 27 is added to it, yield the second number multiplied by itself and this product is multiplied by 9. | ||
| + | :I ask: how much will each number be? | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle a:b=3:4\\\scriptstyle\left(a\sdot b\right)^2+27=9b^2\end{cases}</math> | ||
| + | |style="text-align:right;"|עשה לי זה החשבון תמצא לי שני מספרי' שיהיה הראשון חלק מהשני כמו שג' הוא מד‫'<br> | ||
| + | ומוכה הראשון בשני ואותה ההכאה תוכה בעצמה ויחובר עמה כ"ז יעשה המספר השני בכפלו בעצמו ואותה ההכאה תוכה בט‫'<br> | ||
| + | אשאל כמה הוא כל אחד מהמספרים האמורי‫'{{#annotend:gmur}} | ||
| + | |- | ||
| + | |The procedure: | ||
| + | |style="text-align:right;"|זהו מעשהו | ||
| + | |- | ||
| + | | | ||
| + | :Suppose the first number is three things [<math>\scriptstyle{\color{blue}{a=3x}}</math>] and the second is four things [<math>\scriptstyle{\color{blue}{b=4x}}</math>]. | ||
| + | |style="text-align:right;"|תניח שהמספר ראשון יהיה ג' דברים והשני יהיה ד' דברים | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{\left(3x\sdot4x\right)^2+27=\left(12x^2\right)^2+27=144x^4+27}}</math> | ||
| + | |style="text-align:right;"|עתה תכה הראשון בשני ועולה י"ב צינסי<br> | ||
| + | ואותה ההכאה מוכה בעצמה ועולה קמ"ד צינסי מצינסי<br> | ||
| + | ותוסיף כ"ז ויהיה לך קמ"ד צינסי מצינסי וכ"ז מספרי' ושמרם | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{9\left(4x\right)^2=9\sdot16x^2}}</math> | ||
| + | |style="text-align:right;"|אח"כ תכה המספר השני שהוא ד' דברים בעצמו ועולה י"ו צינסי<br> | ||
| + | וזאת ההכאה תכה בט‫' | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle144x^4+27=144x^2</math> | ||
| + | |style="text-align:right;"|ועולה קמ"ד צינסי אשר יבאו להיות שוים אל החלק האחר אשר שמרת וזהו אל קמ"ד צינסי מצינסי וכ"ז מספרי‫' | ||
| + | |- | ||
| + | | | ||
| + | :<span style=color:Green>'''Normalization''':</span> <math>\scriptstyle{\color{blue}{144x^4+27=144x^2\quad/\div144}}</math> | ||
| + | |style="text-align:right;"|תחלק כל ההשואה על כמות הצינסי מצינסי שזהו על קמ"ד | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle x^4+\frac{27}{144}=x^2</math> | ||
| + | |style="text-align:right;"|ויהיה לך א' צינסו מצינסו וכ"ז מקמ"ד מספרים שוים אל א' צינסו | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle x&\scriptstyle=\sqrt{\frac{1}{2}+\sqrt{\left(\frac{1}{2}\right)^2-\frac{27}{144}}}\\&\scriptstyle=\sqrt{\frac{1}{2}+\sqrt{\frac{1}{4}-\frac{27}{144}}}\\&\scriptstyle=\sqrt{\frac{1}{2}+\sqrt{\frac{9}{144}}}\\\end{align}}}</math> | ||
| + | |style="text-align:right;"|עתה תחלק כמות הצינסו שהוא א' לחצי ויהיה לך חצי אחד<br> | ||
| + | ותכהו בעצמו ועולה א' רביע<br> | ||
| + | ומזה הרביע תוציא המספרים שהם כ"ז מקמ"ד וישאר ט' מקמ"ד<br> | ||
| + | ושרש ט' מקמ"ד תחברם על החצי האחד מהצינסי וזהו על חצי אחד ויהיה לך חצי אחד ושרש ט' מקמ"ד<br> | ||
| + | ושרש זה הסך יבא להיות שוה הדבר | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ואתה הנחת היות המספר ראשון ג' דברי' א"כ | ||
| + | תכה ג' בשרש מחבור שרש ט' מקמ"ד עם חצי<br> | ||
| + | שעולה שרש מחבור שרש מה' וט' מקמ"ד עם ד' וחצי וככה יבא להיות המספר הראשון<br> | ||
| + | שהוא ו' וג' רביעי‫'<br> | ||
| + | מפני כי שרש ה' וט' מקמ"ד הוא ב' ורביע<br> | ||
| + | ומחובר עם ד' וחצי עושה ו' וג' רביעי‫'<br> | ||
| + | ושרש זה הו' וג' רביעי' כאמור יבא להיות המספר הראשון | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | ::<math>\scriptstyle{\color{blue}{a=3x=3\sqrt{\frac{1}{2}+\sqrt{\frac{9}{144}}}=\sqrt{\left(4+\frac{1}{2}\right)+\sqrt{5+\frac{9}{144}}}=\sqrt{\left(4+\frac{1}{2}\right)+\left(2+\frac{1}{4}\right)}=\sqrt{6+\frac{3}{4}}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|והשני הנחת היותו ד' דברים<br> | ||
| + | א"כ תכה ד' בשרש מחבור שרש ט' מקמ"ד עם חצי<br> | ||
| + | ועולה שורש מחבור שרש מי"ו שהוא ד' עם ח' שעולה י"ב<br> | ||
| + | א"כ המספר השני יבא להיות שרש י"ב | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | ::<math>\scriptstyle{\color{blue}{b=4x=4\sdot\sqrt{\frac{1}{2}+\sqrt{\frac{9}{144}}}=\sqrt{8+\sqrt{16}}=\sqrt{8+4}=\sqrt{12}}}</math> | ||
| + | |- | ||
| + | |The answer of this calculation is by the adding the root to half the squares. | ||
| + | |style="text-align:right;"|ודע כי זה החשבון נעשית התשובה באופן השרש ש{{#annot:term|178,1215|wORm}}יתחבר עם{{#annotend:wORm}} חצי הצינסי | ||
| + | |- | ||
| + | |It can be solved also by subtracting the root from half the squares: | ||
| + | |style="text-align:right;"|ואפש' לענותו ג"כ באופן שיוצא השרש ממחצית הצינסי | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|באופן זה שכאשר הוצאת המספרי' מהכאת מחצית הצינסי ישאר לך ט' מקמ"ד<br> | ||
| + | ושרש זה הט' מקמ"ד בתשובה הראשונה חברת על מחצית הצינסי שהיה חצי ונשאר פחות שרש ט' מקמ"ד<br> | ||
| + | ושרש זה השארית יבא להיות הדבר | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | ::<math>\scriptstyle{\color{blue}{x_2=\sqrt{\frac{1}{2}-\sqrt{\left(\frac{1}{2}\right)^2-\frac{27}{144}}}=\sqrt{\frac{1}{2}-\sqrt{\frac{9}{144}}}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|א"כ הדבר אשר הנחת היותו ג' דברים יבא להיות ג' מוכה בשרש ממוצא שרש מט' מקמ"ד חוצה מחצי<br> | ||
| + | שעולה שרש ממוצא שרש מה' וט' מקמ"ד חוצה מד' וחצי וככה יבא להיות המספר הראשון<br> | ||
| + | וזהו שרש ב' וחצי<br> | ||
| + | אשר כאשר הושב אל מספר מדבר יבא להיות א' וחצי וככה הוא המספר ראשון | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | ::<math>\scriptstyle{\color{blue}{a_2=3x_2=3\sqrt{\frac{1}{2}-\sqrt{\frac{9}{144}}}=\sqrt{\left(4+\frac{1}{2}\right)-\sqrt{5+\frac{9}{144}}}=\sqrt{2+\frac{1}{{\color{red}{4}}}}=1+\frac{1}{2}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|והשני הנחת היותו ד' דברים<br> | ||
| + | א"כ תכה ד' בשרש ממוצא שרש מט' מקמ"ד חוץ מחצי<br> | ||
| + | ועולה שרש ממוצא שרש י"ו שהוא ד' חוץ מח' ונשאר ד‫'<br> | ||
| + | ושרש אלו הד' שהוא ב' יבא להיות המספר השני | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | ::<math>\scriptstyle{\color{blue}{b_2=4x_2=4\sqrt{\frac{1}{2}-\sqrt{\frac{9}{144}}}=\sqrt{8-\sqrt{16}}=\sqrt{8-4}=\sqrt{4}=2}}</math> | ||
| + | |- | ||
| + | |So, the problem is solved by both the above mentioned procedures | ||
| + | |style="text-align:right;"|והנה נענה זה החשבון בשני האופנים האמורי' למעלה | ||
| + | |- | ||
| + | |There are problems that can be solved by both procedures, and both are correct, as seen in this problem. | ||
| + | |style="text-align:right;"|שאפש' בחשבונו' מה לענות בשני האופני' באמת כן באחד כמו בשני כאשר הראית בזה החשבון | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ===== Chapter 45 - II ===== | ||
| + | |||
| + | |style="width:45%;text-align:right;"|<big>פרק מ"ה</big> | ||
| + | |- | ||
| + | |There are problems that can be solved only by one of the procedures of the above chapter: | ||
| + | |style="text-align:right;"|עוד רצוני להראותך איך הפרק האמור אי אפש' ליתן תשובה בחשבונות מה רק באחד מהאופני' האמורים כמו שאראך מכאן ולהבא בזה החשבון הנמשך | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:a+b=10, (a-b)²·7⅑=(ab)²|619|qB2c}}Divide ten into two parts, such that when the the difference between them is multiplied by itself and this product is multiplied by 7 and a ninth, it yields the same as the product of the first by the second, then this product is multiplied by itself. | ||
| + | :I ask: how much will each one of the parts be? | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle a+b=10\\\scriptstyle\left(a-b\right)^2\sdot\left(7+\frac{1}{9}\right)=\left(a\sdot b\right)^2\end{cases}</math> | ||
| + | |style="text-align:right;"|עשה לי זה החשבון תחלק עשרה לשני חלקים באופן שמוכה ההבדל שביניהם בעצמו ואותה ההכאה תוכה בז' ותשיעית יעשה כמו מוכה הראשון בשני ואותה ההכאה תוכה בעצמה<br> | ||
| + | אשאל כמה יבא להיות כל אחד מהחלקים{{#annotend:qB2c}} | ||
| + | |- | ||
| + | |The procedure: | ||
| + | |style="text-align:right;"|זהו מעשהו | ||
| + | |- | ||
| + | | | ||
| + | :Suppose one of the parts is a thing plus five [<math>\scriptstyle{\color{blue}{a=x+5}}</math>] and the other is the remainder from ten, which is five minus a thing [<math>\scriptstyle{\color{blue}{b=10-\left(x+5\right)=5-x}}</math>]. | ||
| + | |style="text-align:right;"|תניח שאחד מהחלקים יהיה דבר אחד וחמשה והאחר יבא להיות השארית עד עשרה שהוא ה' פחות דבר אחד | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|עתה תקח ההבדל אשר בין האחד החלק אל האחר אשר יבא להיות ב' דברים<br> | ||
| + | מפני שהחלק הגדול הוא ב' דברים יותר מהשני<br> | ||
| + | ותכה זה ההבדל שהוא ב' דברים בעצמו ויהיה עולה ד' צינסי<br> | ||
| + | וזאת ההכאה תכה בז' ותשיעית וזהו ד' צינסי וז' וא' תשיעית ועולה כ"ח וד' מט' ותשמרם לאחד מחלקי ההשואה | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | :::<math>\scriptstyle{\color{blue}{\left[\left(x+5\right)-\left(5-x\right)\right]^2\sdot\left(7+\frac{1}{9}\right)=\left(2x\right)^2\sdot\left(7+\frac{1}{9}\right)=4x^2\sdot\left(7+\frac{1}{9}\right)=\left(28+\frac{4}{9}\right)x^2}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{\left[\left(x+5\right)\sdot\left(5-x\right)\right]^2=\left(25-x^2\right)^2}}</math> | ||
| + | |style="text-align:right;"|עתה תכה הראשון בשני שזהו דבר אחד וה' בה' פחות דבר אחד ועולה כ"ה מספרי' פחות א' צינסי<br> | ||
| + | ואלו כ"ה מספרים פחות א' צינסו תכם בעצמם | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle x^4+625-50x^2=\left(28+\frac{4}{9}\right)x^2</math> | ||
| + | |style="text-align:right;"|ועולה א' צינסו מצינסו ותרכ"ה מספרי' פחות נ' צינסי שהם שוים אל החלק האחר אשר שמרת שזהו אל כ"ח צינסי וד' תשיעיות | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{x^4+625-50x^2=\left(28+\frac{4}{9}\right)x^2\quad/+50x^2}}</math> | ||
| + | |style="text-align:right;"|עתה תחבר נ' צינסי הפוחת מהחלק האחד אל כל אחד מהחלקים | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle x^4+625=\left({\color{red}{78}}+\frac{4}{9}\right)x^2</math> | ||
| + | |style="text-align:right;"|ויהיה לך א' צינסו מצינסו ותרכ"ה מספרים שוים אל תשפ"ט צינסי וד' מט‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Pursuing the above rule: | ||
| + | |style="text-align:right;"|עתה תרדוף כפי הכלל האמור למעלה | ||
| + | |- | ||
| + | | | ||
| + | :<span style=color:Green>'''Normalization''':</span> <math>\scriptstyle{\color{blue}{x^4+625=\left({\color{red}{78}}+\frac{4}{9}\right)x^2\quad/\div1}}</math> | ||
| + | |style="text-align:right;"|תחלק כל ההשואה על כמות הצינסי מצינסי שזהו א' ויהיה לך אותו בעצמו | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|אח"כ תחלק כמות הצינסי לחצי שזהו ע"ח וד' תשיעיות ויעלה מזה ל"ט וב' מט‫' | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ואלו הל"ט וב' מט' תכה בעצמם ועולה אלף תקל"ח ול"א מפ"א | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ומזאת ההכאה תוציא המספרים שהם תרכ"ה וישאר תתקי"ג ול"א מפ"א | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|ושרש זה הנשאר {{#annot:term|181,1232|ls9T}}תוציא חוצה מ{{#annotend:ls9T}}החצי האחד מהצינסי שזהו חוץ מל"ט וב' מט‫'<br> | ||
| + | ויהיה לך ל"ט וב' מט' פחות שרש תתקי"ג ול"א מפ"א ושרש זה הנשאר יבא לשוות הדבר | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | ::<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle x&\scriptstyle=\sqrt{\left[\frac{1}{2}\sdot\left(78+\frac{4}{9}\right)\right]-\sqrt{\left[\frac{1}{2}\sdot\left(78+\frac{4}{9}\right)\right]^2-625}}=\sqrt{\left(39+\frac{2}{9}\right)-\sqrt{\left(39+\frac{2}{9}\right)^2-625}}\\&\scriptstyle=\sqrt{\left(39+\frac{2}{9}\right)-\sqrt{\left(1538+\frac{31}{81}\right)-625}}=\sqrt{\left(39+\frac{2}{9}\right)-\sqrt{913+\frac{31}{81}}}\\\end{align}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{a=x+5=\sqrt{\left(39+\frac{2}{9}\right)-\sqrt{913+\frac{31}{81}}}+5}}</math> | ||
| + | |style="text-align:right;"|ואתה הנחת שאחד מהחלקים היה דבר אחד וה‫'<br> | ||
| + | א"כ יבא להיות החלק הראשון ה' ומוצא שרש מתתקי"ג ול"א מפ"א חוץ מל"ט וב' מט‫'<br> | ||
| + | ושרש זה הנשאר אוסף על אותו הה' אשר הנחת אחד מהחלקים היותו דבר אחד וה' יבא להיות החלק הראשון | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{b=5-x=5-\sqrt{\left(39+\frac{2}{9}\right)-\sqrt{913+\frac{31}{81}}}}}</math> | ||
| + | |style="text-align:right;"|והחלק האחר יבא להיות ה' פחות הדבר האמור<br> | ||
| + | שהוא פחות שרש ממוצא שרש מתתקי"ג ול"א מפ"א חוץ מל"ט וב' מט' וככה יבא להיות החלק האחר מעשרה | ||
| + | |- | ||
| + | |This is solved only by one of the solving procedures mentioned above, which is by subtracting: | ||
| + | |style="text-align:right;"|עתה אזכירך שהדבר נענה באופן אחד מהאופנים שהוא באופן מההוצאה האמור קודם בכלל האמור בפרק האמור | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|שהוא שהדבר יבא להיות שרש ממוצא מתתקי"ג ול"א מפ"א שהוא ל' וב' מט‫'<br> | ||
| + | חוץ מל"ט וב' מט' וישאר ט‫'<br> | ||
| + | ושרש זה השארית שהוא ט' הנה הוא ג‫'<br> | ||
| + | יבא להיות הדבר א"כ יבא | ||
| + | להיות החלק הראשון שהוא א' דבר וה' ג' וה' יעלה ח' וככה הוא החלק הראשון | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | ::<math>\scriptstyle{\color{blue}{a=x+5=\sqrt{\left(39+\frac{2}{9}\right)-\sqrt{913+\frac{31}{81}}}+5=\sqrt{\left(39+\frac{2}{9}\right)-\left(30+\frac{2}{9}\right)}+5=\sqrt{9}+5=3+5=8}}</math> | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{b=5-x=5-3=2}}</math> | ||
| + | |style="text-align:right;"|והחלק השני הוא ה' פחות דבר אחד והדבר הוא ג' שישאר ב' וכן הוא החלק השני | ||
| + | |- | ||
| + | |It cannot be solved by the other procedure of the above chapter. | ||
| + | |style="text-align:right;"|ובאופן אחר אי אפש' לענות הדבר בהשיב זה החשבון אל זה הפרק | ||
| + | |- | ||
| + | |Since one of the part is <math>\scriptstyle{\color{blue}{x+5}}</math> | ||
| + | |style="text-align:right;"|מפני כי אחד מהחלקים יבא להיות דבר אחד וה‫' | ||
| + | |- | ||
| + | | | ||
| + | :<math>\scriptstyle x=\sqrt{\left(\frac{1}{2}\sdot\frac{b}{a}\right)+\sqrt{\left(\frac{1}{2}\sdot\frac{b}{a}\right)^2-\frac{c}{a}}}>5</math> | ||
| + | |style="text-align:right;"|ובזולת אותו השרש מהנשאר בהוצאת המספרי' מהכאת מחצית כמות הצינסי לבד השרש ממחצית כמות הצינסי יבא להיות יותר מה‫' | ||
| + | |- | ||
| + | | | ||
| + | :<math>\scriptstyle x+5>10</math> | ||
| + | |style="text-align:right;"|א"כ יבא להיו' אחד מהחלקי' ה' ודבר אחד והדבר יבא להיות יותר מה' באופן שהחלק האמור יבא להיות יותר מעשרה | ||
| + | |- | ||
| + | |and it is impossible that the part will be greater than the whole | ||
| + | |style="text-align:right;"|וזה דבר נמנע שהחלק יהיה גדול מהכל | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ===== Chapter 45 - III ===== | ||
| + | |||
| + | |style="width:45%;text-align:right;"|<big>פרק מ"ה</big> | ||
| + | |- | ||
| + | |Sometimes the answer is given only by the other procedure of the above chapter, i.e. the root of the sum. | ||
| + | |style="text-align:right;"|עוד רצוני להשים לפניך חשבון אחר אשר עמו יראה כי הפרק האמור יתן תשובה לפעמים היות הדבר לבד באופן האמור קודם רצו' שרש החבור | ||
| + | |- | ||
| + | |Example: | ||
| + | |style="text-align:right;"|ו{{#annot:term|898,1896|FaM1}}אתן לך המשל{{#annotend:FaM1}} פה בקרוב באופן זה כאמור | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:a+b=10, (ab+a²)a=(a½a)²+36|619|HXRY}}Divide ten into two parts, such that when the larger is multiplied by the smaller and [the product] is added to the product of the larger by itself, then the sum is multiplied by the larger, it yields the same as the product of the larger by its half, then this product is multiplied by itself and 36 is added to it. | ||
| + | :I ask: how much will each one of the parts be? | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle a+b=10\\\scriptstyle\left[\left(a\sdot b\right)+a^2\right]\sdot a=\left[a\sdot\left(\frac{1}{2}a\right)\right]^2+36\end{cases}</math> | ||
| + | |style="text-align:right;"|עשה לי מעשרה ב' חלקים באופן שבהכות הגדול בקטן ויחובר אל הכאת הגדול בעצמו ואותו הסך יוכה בחלק הגדול יעשה כמו הכאת הגדול בחציו ואותה ההכאה תוכה בעצמה ויחובר אליה ל"ו<br> | ||
| + | אשאל כמה יבא להיות כל אחד מהחלקי‫'{{#annotend:HXRY}} | ||
| + | |- | ||
| + | |The procedure: | ||
| + | |style="text-align:right;"|זו היא {{#annot:term|469|S30z}}פעולתו{{#annotend:S30z}} | ||
| + | |- | ||
| + | | | ||
| + | :Suppose the larger part is a thing [<math>\scriptstyle{\color{blue}{a=x}}</math>] and the smaller is the remainder from ten, which is ten minus a thing [<math>\scriptstyle{\color{blue}{b=10-x}}</math>]. | ||
| + | |style="text-align:right;"|תניח שהחלק הגדול יהיה דבר אחד והקטן יבא להיות הנשאר עד עשרה שהוא עשרה פחות דבר | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{x\sdot\left(10-x\right)=10x-x^2}}</math> | ||
| + | |style="text-align:right;"|עתה תכה הגדול בקטן רצו' דבר אחד בעשרה פחות דבר אחד ועולה י' דברי' פחות א' צינסו | ||
| + | |- | ||
| + | | | ||
| + | ::::<math>\scriptstyle{\color{blue}{\left(10x-x^2\right)+\left(x\sdot x\right)=10x-x^2+x^2=10x}}</math> | ||
| + | |style="text-align:right;"|ועל זה תחבר הכאת הגדול המוכה בעצמו שהוא דבר אחד בדבר אחד ועולה א' צינסו ויהיה לך בסך עשרה דברים | ||
| + | |- | ||
| + | | | ||
| + | :::::<math>\scriptstyle{\color{blue}{10x\sdot x=10x^2}}</math> | ||
| + | |style="text-align:right;"|ותכה זה הסך שהוא י' דברי' בחלק הגדול שהוא דבר אחד ועולה י' צינסי ושמרם לחלק אחד מההשואה | ||
| + | |- | ||
| + | | | ||
| + | :::<math>\scriptstyle{\color{blue}{x\sdot\left(\frac{1}{2}x\right)=\frac{1}{2}x^2}}</math> | ||
| + | |style="text-align:right;"|אח"כ תכה הגדול שהוא א' דבר בחציו שהוא חצי דבר ועולה חצי צינסו | ||
| + | |- | ||
| + | | | ||
| + | ::::<math>\scriptstyle{\color{blue}{\left(\frac{1}{2}x^2\right)^2}}</math> | ||
| + | |style="text-align:right;"|וזאת ההכאה רצוני חצי צינסו תכה בעצמה | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle\frac{1}{4}x^4+36=10x^2</math> | ||
| + | |style="text-align:right;"|ועולה א' רביע מצינסו מצינסו ול"ו מספרי' להיות שוים אל עשרה צינסי אשר שמרת | ||
| + | |- | ||
| + | | | ||
| + | ::Pursuing the above rule: | ||
| + | |style="text-align:right;"|עתה תרדוף כפי הכלל האמור למעלה | ||
| + | |- | ||
| + | | | ||
| + | :<span style=color:Green>'''Normalization''':</span> <math>\scriptstyle{\color{blue}{\frac{1}{4}x^4+36=10x^2\quad/\div\frac{1}{4}}}</math> | ||
| + | |style="text-align:right;"|תחלק כל ההשוא' בכמות הצינסי מצינסי שהוא א' רביע | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle x^4+144=40x^2</math> | ||
| + | |style="text-align:right;"|ויהיה לך א' צינסו מצינסו וקמ"ד מספרי' שוים אל מ' צינסי | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|אח"כ תחלק כמות הצינסי שהם מ' לחצאי' ויבא מזה כ‫'<br> | ||
| + | ואלו הכ' תכם בעצמם ועולה ת‫'<br> | ||
| + | ומאלו הת' תוציא המספרי' שהם קמ"ד וישאר רנ"ו<br> | ||
| + | ושרש אלו הרנ"ו תחבר על החצי האחר מהצינסי שזהו על כ' ויהיה לך כ' ושרש רנ"ו<br> | ||
| + | ושרש זה הסך יבא להיות הדבר<br> | ||
| + | וכן הוא החלק הגדול אשר הנחת היותו דבר אחד | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | ::<math>\scriptstyle{\color{blue}{a=x=\sqrt{\left(\frac{1}{2}\sdot40\right)+\sqrt{\left(\frac{1}{2}\sdot40\right)^2-144}}=\sqrt{20+\sqrt{20^2-144}}=\sqrt{20+\sqrt{400-144}}=\sqrt{20+\sqrt{256}}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{b=10-x=10-\sqrt{20+\sqrt{256}}}}</math> | ||
| + | |style="text-align:right;"|והחלק האחר יבא להיות הנשאר עד י‫'<br> | ||
| + | וזהו עשרה פחות שרש מחבור שרש רנ"ו על הכ' שהם מחצית כמות הצינסי<br> | ||
| + | וככה יבא להיות החלק הקטן מעשרה | ||
| + | |- | ||
| + | |It is solved by the first procedure of the [above] chapter, i.e. the root of the sum, but it cannot be solved by the other procedure. | ||
| + | |style="text-align:right;"|ונענה באופן ראשון שמשים הפרק שזהו שרש החבור ולא יתכן תשובתו באחד מהאופני' האחרי‫' | ||
| + | |- | ||
| + | | | ||
| + | :The reason is that | ||
| + | :<math>\scriptstyle{\color{blue}{a_2=x_2=\sqrt{20-\sqrt{256}}<\frac{1}{2}\sdot10}}</math> | ||
| + | |style="text-align:right;"|וסבת למה זה היא כי כשהוצא שרש רנ"ו ממחצית הצינסי שזהו הכ' שרש הנשאר יבא להיות פחות ממחצית עשרה | ||
| + | |- | ||
| + | | | ||
| + | :But, <math>\scriptstyle{\color{blue}{a=x}}</math> is the larger of the two parts of 10. | ||
| + | |style="text-align:right;"|וכבר אמרנו קודם שהחלק הגדול היה דבר אחד ולעשות מעשרה שני חלקי‫' | ||
| + | |- | ||
| + | | | ||
| + | :It is impossible that the larger part of 10 will be greater than 5 as well as less than 5 | ||
| + | |style="text-align:right;"|ולקחת הגדול הנה הגדול יבא להיות יותר מה' וכפי זה החשבון היה בא החלק הגדול פחו' מה' וזהו דבר נמנע שיהיה החלק מי' פחות מה‫' | ||
| + | |- | ||
| + | |Therefore: | ||
| + | ::<math>\scriptstyle{\color{blue}{a=x=\sqrt{20+\sqrt{256}}=\sqrt{20+16}=\sqrt{36}=6}}</math> | ||
| + | |style="text-align:right;"|א"כ תחבר שרש רנ"ו שהוא י"ו על כ' עולה ל"ו<br> | ||
| + | ושרש זה הסך מל"ו שיבא להיות ו' הוא החלק ראשון אשר הונח היותו דבר אחד | ||
| + | |- | ||
| + | | | ||
| + | ::<math>\scriptstyle{\color{blue}{b=10-x=4}}</math> | ||
| + | |style="text-align:right;"|והחלק הקטן יבא להיות הנשאר עד י' שהוא ד‫' | ||
| + | |- | ||
| + | |The reason that the larger part was given as <math>\scriptstyle{\color{blue}{a=x}}</math>, was to solve it by the third way of the above chapter, i.e. to show that sometimes it is impossible to solve by the other procedure, only by the root of the sum. | ||
| + | |style="text-align:right;"|עתה דע כי הסבה שהנחנו החלק הגדול דבר א' היתה כדי להשיב תשובת זה הפרק אל התשוב' השלישית אשר הונחה בראשית הפרק ולהראות כי בפרקי' מה הדבר אי אפש' לענות באופן אחר כי אם שרש הסך כאשר אמרנו קודם | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== Chapter 46 ==== | ||
| + | |||
| + | |style="width:45%;text-align:right;"|<big>פרק מ"ו</big> | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|עוד רצוני לרדוף וזהו הוא בזה האופן | ||
| + | |- | ||
| + | |{{#annot:ax⁴=bx²+c|719|CLbn}}When squares of squares are equal to a number and squares: | ||
| + | :<math>\scriptstyle ax^4=bx^2+c</math> | ||
| + | |style="text-align:right;"|כאשר הצינסי מצינסי יהיו שוים אל המספר והצינסי{{#annotend:CLbn}} | ||
| + | |- | ||
| + | |The whole equation should be divided by the number of the squares of squares. | ||
| + | |style="text-align:right;"|צריך לחלק כל ההשואה על כמות הצינסי מצינסי | ||
| + | |- | ||
| + | |Then, the number of the squares should be divided in half. | ||
| + | |style="text-align:right;"|ואח"כ לחלק כמות הצינסי לחצאי‫' | ||
| + | |- | ||
| + | |Each of these halves is divided by itself. | ||
| + | |style="text-align:right;"|ולהכות אחד מהחצאי' ההם בעצמו | ||
| + | |- | ||
| + | |Add the numbers to this product. | ||
| + | |style="text-align:right;"|ועל זאת ההכא' תחבר המספרי‫' | ||
| + | |- | ||
| + | |Add the root of this sum to the other half of the number of squares. | ||
| + | |style="text-align:right;"|ושרש זה הסך תחבר על החצי האחר מכמות הצינסי | ||
| + | |- | ||
| + | |The root of this final sum is equal to the thing. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{x=\sqrt{\sqrt{\left(\frac{1}{2}\sdot\frac{b}{a}\right)^2+\frac{c}{a}}+\left(\frac{1}{2}\sdot\frac{b}{a}\right)}}}</math> | ||
| + | |style="text-align:right;"|ושרש זה הסך האחרון יבא לשוו' הדבר | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:a²=2b+8;a²+b²=80|618|Ob3R}}Find me two numbers such that the one multiplied by itself yields 2 times the second and 8 more; | ||
| + | :and when each of them is multiplied by itself, and the two products are summed together, they yield 80. | ||
| + | :I ask: how much will each number be? | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle a^2=2b+8\\\scriptstyle a^2+b^2=80\end{cases}</math> | ||
| + | |style="text-align:right;"|והנה המשל עשה לי זה החשבון תמצא לי ב' מספרי' שכאשר יוכה הראשון בעצמו יעשה ב' דמיוני השני וח' יותר<br> | ||
| + | ומוכה כל אחד מהם בעצמו ויקובצו ב' ההכאו' יחד יעשו פ‫'<br> | ||
| + | אשאל כמה יבא להיות כל אחד מהמספרי‫'{{#annotend:Ob3R}} | ||
| + | |- | ||
| + | | | ||
| + | :Suppose the first number is a thing [<math>\scriptstyle{\color{blue}{a=x}}</math>]. | ||
| + | |style="text-align:right;"|תניח שהמספר הראשון יהיה דבר אחד | ||
| + | |- | ||
| + | | | ||
| + | :Multiply it by itself the result is 1 square. | ||
| + | :<math>\scriptstyle{\color{blue}{a^2=x^2}}</math> | ||
| + | |style="text-align:right;"|וזה תכהו בעצמו ועולה א' צינסו | ||
| + | |- | ||
| + | | | ||
| + | :The second number then is half a square minus four [<math>\scriptstyle{\color{blue}{b=\frac{1}{2}x^2-4}}</math>]. | ||
| + | |style="text-align:right;"|א"כ יבא להיות המספר השני חצי צינסו פחות ד‫' | ||
| + | |- | ||
| + | | | ||
| + | :Multiply each of them by itself: | ||
| + | |style="text-align:right;"|עתה תכה כל אחד מהם בעצמו | ||
| + | |- | ||
| + | | | ||
| + | :The first, which is one thing; the result is 1 square. | ||
| + | :<math>\scriptstyle{\color{blue}{x\sdot x=x^2}}</math> | ||
| + | |style="text-align:right;"|שהם הראשון שהוא דבר אחד ועולה א' צינסו | ||
| + | |- | ||
| + | | | ||
| + | :The second, which is half a square minus 4, by itself; the result is a quarter of a square of a square and 16 numbers minus 4 squares. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(\frac{1}{2}x^2-4\right)^2=\frac{1}{4}x^4+16-4x^2}}</math> | ||
| + | |style="text-align:right;"|והשני שהוא חצי צינסו פחות ד' בעצמו ועולה א' רביע מצינסו מצינסו וי"ו מספרי' פחות ד' צינסו | ||
| + | |- | ||
| + | | | ||
| + | :Sum up these two products together: | ||
| + | |style="text-align:right;"|וקבץ ב' אלו ההכאו' יחד | ||
| + | |- | ||
| + | | | ||
| + | :It is 1 square and a quarter of a square of a square plus 16 numbers minus 4 squares. | ||
| + | :<math>\scriptstyle{\color{blue}{x^2+\left(\frac{1}{4}x^4+16-4x^2\right)}}</math> | ||
| + | |style="text-align:right;"|וזהו א' צינסו עם רביע צינסו מצינסו וי"ו מספרי' פחות ד' צינסי | ||
| + | |- | ||
| + | | | ||
| + | :The result is a quarter of a square of a square and 16 numbers minus 3 squares equal 80. | ||
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{4}x^4+16-3x^2=80}}</math> | ||
| + | |style="text-align:right;"|עולה א' רביע צינסו מצינסו וי"ו מספרי' פחות ג' צינסי שהם שוים אל פ‫' | ||
| + | |- | ||
| + | | | ||
| + | :Pursue the above given rule: | ||
| + | |style="text-align:right;"|עתה תרדוף כפי הכלל הנתון למעלה | ||
| + | |- | ||
| + | | | ||
| + | :<span style=color:Green>'''Normalization''':</span> Divide the whole equation by the number of the squares of squares, that is by a quarter. | ||
| + | |style="text-align:right;"|תחלק כל ההשואה על כמו' הצינסי מצינסי וזהו על א' ורביע | ||
| + | |- | ||
| + | | | ||
| + | :<span style=color:Green>'''Restoration''':</span> But, before you divide this equation, add the subtractive part, which is 3 squares, to each of the sides and subtract the smaller number from each of the sides. | ||
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{4}x^4+16-16-3x^3+3x^3=80-16+3x^3}}</math> | ||
| + | |style="text-align:right;"|וקודם שתחלק זאת ההשוא' תתן לאשר לו פחות א' מהחלקי' שהוא ג' צינסי אל כל א' מהחלקי‫'<br> | ||
| + | והוצא הכמות הקטן מהמספרי' מכל א' מהחלקי‫' | ||
| + | |- | ||
| + | | | ||
| + | :You are left with a reduced equation: a quarter of a square of a square equals 3 squares plus 64 numbers. | ||
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{4}x^4=3x^3+64}}</math> | ||
| + | |style="text-align:right;"|וישאר לך ההשוא' נקיה א' ורביע צינסו מצינסו שוה אל ג' צנסי ואל ס"ד מספרי‫' | ||
| + | |- | ||
| + | | | ||
| + | :<span style=color:Green>'''Normalization''':</span> Divide by a quarter. | ||
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{4}x^4=3x^3+64\quad/\div\frac{1}{4}}}</math> | ||
| + | |style="text-align:right;"|לחלק על א' רביע | ||
| + | |- | ||
| + | | | ||
| + | :The result is 1 square of a square equals 12 squares plus 256 numbers. | ||
| + | :<math>\scriptstyle{\color{blue}{x^4=12x^3+256}}</math> | ||
| + | |style="text-align:right;"|ויבא מזה א' צינסו מצינסו שוה אל י"ב צינסי ורנ"ו מספרי‫' | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|אח"כ תחלק כמות הצינסי שהם י"ב לחצי ויבא ו‫'<br> | ||
| + | ואלו הו' תכם בעצמם ועולה ל"ו<br> | ||
| + | ועל אלו הל"ו תחבר המספרי' שהם רנ"ו ועולה רצ"ב<br> | ||
| + | ושרש אלו הרצ"ב תחבר על החצי האחד מהצינסי שהוא ו' ויהיה לך ו' ושרש רצ"ב<br> | ||
| + | ושרש זה הסך יבא להיות הדבר וכן הוא המספר הראשון | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | ::<math>\scriptstyle{\color{blue}{a=x=\sqrt{\sqrt{\left(\frac{1}{2}\sdot12\right)^2+256}+\left(\frac{1}{2}\sdot12\right)}=\sqrt{\sqrt{6^2+256}+6}=\sqrt{\sqrt{36+256}+6}=\sqrt{\sqrt{292}+6}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|עתה תכה זה שיבא להיות הדבר בעצמו ויהיה לך ו' ושרש רצ"ב<br> | ||
| + | ומזה הסך תוציא ח' וישאר שרש מרצ"ב פחות ב' אשר יבא להיות כפל למספר השני<br> | ||
| + | א"כ תחלק זה השארית לחצי שהוא ב' ועולה מזה שרש מע"ג פחות אחד וככה יבא להיות המספר השני | ||
| + | |- | ||
| + | |colspan=2| | ||
| + | ::<math>\scriptstyle{\color{blue}{b=\frac{1}{2}x^2-4=\frac{1}{2}\left(\sqrt{\sqrt{292}+6}\right)^2-4=\frac{1}{2}\sdot\left(\sqrt{292}+6-8\right)=\frac{1}{2}\sdot\left(\sqrt{292}-2\right)=\sqrt{73}-1}}</math> | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== Chapter 47 ==== | ||
| + | |||
| + | |style="width:45%;text-align:right;"|<big>פרק מ"ז</big> | ||
| + | |- | ||
| + | |{{#annot:bx=³√c|726|NHZd}}When things are equal to a cube root of the numbers: | ||
| + | :<math>\scriptstyle bx=\sqrt[3]{c}</math> | ||
| + | |style="text-align:right;"|כאשר הדברי' יהיו שוי' אל שרש מעוק' ממספרי‫'{{#annotend:NHZd}} | ||
| + | |- | ||
| + | |The number of the things should be cubed. | ||
| + | |style="text-align:right;"|צריך להשיב כמות הדברי' אל מעוק‫' | ||
| + | |- | ||
| + | |Then, the numbers that are the radicand of the cube root should be divided by the cubed number of the things. | ||
| + | |style="text-align:right;"|ואח"כ לחלק המספרי' הנקובי' להיות להם שרש מעוק' על השבת כמות הדברי‫' | ||
| + | |- | ||
| + | |Extract the cube root of the result and it is equal to the thing. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{x=\sqrt[3]{\frac{c}{b^3}}}}</math> | ||
| + | |style="text-align:right;"|ומהעולה {{#annot:term|881,1375|Ys8Q}}תקח שרשו המעו'{{#annotend:Ys8Q}} יבא לשוות הדבר | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:a/b=⅔;3a+4b=³√216|618|w4Ac}}Find me two numbers such that one is a part of the other as 2 is of 3; | ||
| + | :and when the first is multiplied by 3 and the second by 4, and both products are summed together, they yield a cube root of 216. | ||
| + | :I ask: how much will each number be? | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle a:b=2:3\\\scriptstyle3a+4b=\sqrt[3]{216}\end{cases}</math> | ||
| + | |style="text-align:right;"|והנה המשל תמצא לי ב' מספרי' שיהיה האחד חלק מהאחר כמו שב' הוא מג‫'<br> | ||
| + | ומוכה הראשון בג' והשני בד' ויחוברו ב' אלו ההכאו' יחד יעשה שרש מעוק' מרי"ו<br> | ||
| + | אשאל כמה יבא להיות כל א' מהמספרי‫'{{#annotend:w4Ac}} | ||
| + | |- | ||
| + | | | ||
| + | :Follow its rule: | ||
| + | |style="text-align:right;"|תעשה כמו שאומ' הכלל שלו | ||
| + | |- | ||
| + | | | ||
| + | :Suppose the first number is two things [<math>\scriptstyle{\color{blue}{a=2x}}</math>] and the second is three [things] [<math>\scriptstyle{\color{blue}{b=3x}}</math>]. | ||
| + | |style="text-align:right;"|תניח שהמספר הראשון יהיה ב' דברי' והשני ג‫' | ||
| + | |- | ||
| + | | | ||
| + | :Now, multiply the first, which is 2 things, by 3; the result is 6. | ||
| + | :<math>\scriptstyle{\color{blue}{2x\sdot3=6x}}</math> | ||
| + | |style="text-align:right;"|עתה תכה הראשון שהוא ב' דברי' בג' ועולה ו‫' | ||
| + | |- | ||
| + | | | ||
| + | :Then, multiply the second, which is 3 things, by 4; the result is 12. | ||
| + | :<math>\scriptstyle{\color{blue}{3x\sdot4=12x}}</math> | ||
| + | |style="text-align:right;"|אח"כ תכה השני שהוא ג' דברי' בד' ועולה י"ב דברי‫' | ||
| + | |- | ||
| + | | | ||
| + | :Sum up both products together, meaning 6 things with 12 things; the result is 18 things that are equal to a cube root of 216. | ||
| + | :<math>\scriptstyle{\color{blue}{6x+12x=18x=\sqrt[3]{216}}}</math> | ||
| + | |style="text-align:right;"|ותקבץ שתי אלו ההכאו' יחד רצו' ו' דברי' עם י"ב דברי' ועולה י"ח דברי' שהם שוים אל שרש מעוק' מרי"ו | ||
| + | |- | ||
| + | | | ||
| + | :Pursue the above given rule: | ||
| + | |style="text-align:right;"|עתה תרדוף כפי הכלל הנתון למעלה | ||
| + | |- | ||
| + | | | ||
| + | :Cube the number of the things, which is 18, this way: multiply 18 by itself; the result is 324. Multiply the 324 by 18; the result is 5832. | ||
| + | |style="text-align:right;"|תשיב כמות הדברי' אל מעוק' וזהו י"ח בזה האופן תכה י"ח בעצמו ועולה שכ"ד ואלו השכ"ד תכם בי"ח ועולה ה' אלפי' ותתל"ב | ||
| + | |- | ||
| + | | | ||
| + | :Divide the number that is the radicand of the cube root, which is 216, by 5832; the result is 1 [part] of [27]. | ||
| + | |style="text-align:right;"|ותחלק כמות המספרי' אשר להם שרש מעו' שהם רי"ו כ"ה אלפי' ותתל"ב ויבא מזה א' מס‫' | ||
| + | |- | ||
| + | | | ||
| + | :The cube root of 1 [part] of 27 is the thing. | ||
| + | :<math>\scriptstyle{\color{blue}{x=\sqrt[3]{\frac{216}{18^3}}=\sqrt[3]{\frac{216}{18^2\sdot18}}=\sqrt[3]{\frac{216}{324\sdot18}}=\sqrt[3]{\frac{216}{5832}}=\sqrt[3]{\frac{1}{27}}}}</math> | ||
| + | |style="text-align:right;"|ושרש מעו' מא' מכ"ז יבא להיות הדבר | ||
| + | |- | ||
| + | | | ||
| + | :You assumed the first number is 2 things, so multiply 2 by the cube root of 1 [part] of 27; the result is a cube root of 8 [parts] of 27, which is 2-thirds, and this is the first number. | ||
| + | :<math>\scriptstyle{\color{blue}{a=2x=2\sdot\sqrt[3]{\frac{1}{27}}=\sqrt[3]{\frac{8}{27}}=\frac{2}{3}}}</math> | ||
| + | |style="text-align:right;"|ואתה הנחת היות המספר הראשון ב' דברי‫'<br> | ||
| + | א"כ תכה ב' בשרש מעו' מא' מכ"ז ועולה שרש מעו' מח' מכ"ז אשר יבא להיות ב' שלישים וככה יבא להיות המספר הראשו‫' | ||
| + | |- | ||
| + | | | ||
| + | :You assumed the second is 3 things, so multiply 3 by the cube root of 1 [part] of 27; the result is a cube root of [27 parts] of 27, which is one integer, 1, and this is the second number. | ||
| + | :<math>\scriptstyle{\color{blue}{b=3x=3\sdot\sqrt[3]{\frac{1}{27}}=\sqrt[3]{\frac{27}{27}}=\sqrt[3]{1}=1}}</math> | ||
| + | |style="text-align:right;"|והשני הנחת היותו ג' דברי‫'<br> | ||
| + | א"כ תכה ג' בשרש מעו' מא' מכ"ז ועלה שרש מכ"ז שהוא אחד שלם שהוא א' וכך יהיה המספר השני | ||
| + | |- | ||
| + | |[The equation] can be returned to chapter 7, by cubing each [part of the equation] by itself. | ||
| + | |style="text-align:right;"|ואפשר להשיב אל פרק ז' בהכות כל אחד בעצמו באופן מעו‫' | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== Chapter 48 ==== | ||
| + | |||
| + | |style="width:45%;text-align:right;"|<big>פרק מ"ח</big> | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|עוד רצו' לשים לפניך חשבון אחר באופן אחר ואומ' כן | ||
| + | |- | ||
| + | |{{#annot:c=³√bx|726|jGFm}}When numbers are equal to a cube root of a thing: | ||
| + | :<math>\scriptstyle c=\sqrt[3]{bx}</math> | ||
| + | |style="text-align:right;"|כאשר המספרי' יהיו שוים אל שרש מעו' מדבר{{#annotend:jGFm}} | ||
| + | |- | ||
| + | |The numbers should be cubed. | ||
| + | |style="text-align:right;"|צריך להשיב כמות המספרי' אל מעו‫' | ||
| + | |- | ||
| + | |Then the cubed [numbers] should be divided by the number of the things that are the radicand of the cube root. | ||
| + | |style="text-align:right;"|ואח"כ לחלק אותה ה{{#annot:term|1071,1430|G4lq}}השבה{{#annotend:G4lq}} על כמות הדברי' הנקובי' להיות להם שרש מעו‫' | ||
| + | |- | ||
| + | | | ||
| + | :The result is equal to the thing. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{x=\frac{c^3}{b}}}</math> | ||
| + | |style="text-align:right;"|ומה שיבא מזה שוה הדבר | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:a/b=¾;³√(2a+3b)=8|618|q0Kk}}Find me two numbers such that one is a part of the other as 3 is of 4; | ||
| + | :and when the one is multiplied by 2 and the other is multiplied by 3, and the two products are summed together their cube root is 8. | ||
| + | :I ask: how much will each number be? | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle a:b=3:4\\\scriptstyle\sqrt[3]{2a+3b}=8\end{cases}</math> | ||
| + | |style="text-align:right;"|והנה המשל עשה לי זה החשבון תמצא לי ב' מספרי‫' | ||
| + | שיהיה הא' חלק מהאחר כמו שג' הוא מד‫'<br> | ||
| + | ומוכה הראשון בב' והשני בג' ויקובצו ב' אלו ההכאו' יחד יהיה שרשם המעוקב ח‫'<br> | ||
| + | אשאל כמה יבא להיות כל א' מהמספרי‫'{{#annotend:q0Kk}} | ||
| + | |- | ||
| + | | | ||
| + | :This is its procedure: | ||
| + | |style="text-align:right;"|זו היא פעולתו | ||
| + | |- | ||
| + | | | ||
| + | :Suppose the first number is three things [<math>\scriptstyle{\color{blue}{a=3x}}</math>] and the second is four [things] [<math>\scriptstyle{\color{blue}{b=4x}}</math>]. | ||
| + | |style="text-align:right;"|תניח שהמספר הראשון יהיה ג' דברי' והשני יהיה ד‫' | ||
| + | |- | ||
| + | | | ||
| + | :Now, multiply the first, which is 3, by 2; the result is 6 things. | ||
| + | :<math>\scriptstyle{\color{blue}{3x\sdot2=6x}}</math> | ||
| + | |style="text-align:right;"|עתה תכה הראשון שהוא ג' בב' עולה ו' דברי‫' | ||
| + | |- | ||
| + | | | ||
| + | :Multiply the second, which is 4 things, by 3; the result is 12. | ||
| + | :<math>\scriptstyle{\color{blue}{4x\sdot3=12}}</math> | ||
| + | |style="text-align:right;"|והשני שהוא ד' דברי' תכה בג' ועולה י"ב | ||
| + | |- | ||
| + | | | ||
| + | :Sum up both products together, which are 6 things with 12 things; the result is 18 things. | ||
| + | :<math>\scriptstyle{\color{blue}{6x+12x=18x}}</math> | ||
| + | |style="text-align:right;"|ותקבץ שתי אלו הכאות יחד שהם ו' דברי' עם י"ב דברי' ועולה י"ח דברי‫' | ||
| + | |- | ||
| + | | | ||
| + | :The cube root of these 18 things is equal to 8. | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt[3]{18x}=8}}</math> | ||
| + | |style="text-align:right;"|ושרש מעו' מאלו הי"ח דברי' יבא להיות שוה אל ח‫' | ||
| + | |- | ||
| + | | | ||
| + | :Pursue the above mentioned rule: | ||
| + | |style="text-align:right;"|עתה תרדוף כפי הכלל האמור למעלה | ||
| + | |- | ||
| + | | | ||
| + | :You should cube the [number]; you get a cubed 8, which is 512. | ||
| + | |style="text-align:right;"|אתה צריך להשיב כמות הדברי' אל מעו' ויהיה לך ח' מושב והוא תקי"ב | ||
| + | |- | ||
| + | | | ||
| + | :Divide this 512 by the number of the things that are the radicand of the cube root, i.e. by 18; the result is 28 and 4 [parts] of 9 and so is the thing. | ||
| + | :<math>\scriptstyle{\color{blue}{x=\frac{8^3}{18}=\frac{512}{18}=28+\frac{4}{9}}}</math> | ||
| + | |style="text-align:right;"|ותחלק אלו התקי"ב בכמות הדברי' הנקובי' להיות להם שרש מעו' וזהו על י"ח ויבא מזה כ"ח וד' מט' וכך יבא לשוות הדבר | ||
| + | |- | ||
| + | | | ||
| + | :You assumed the first number is 3 things, so multiply 3 by 28 and 4 [parts] of 9; the result is 85 and a third and this is the first number. | ||
| + | :<math>\scriptstyle{\color{blue}{a=3x=3\sdot\left(28+\frac{4}{9}\right)=85+\frac{1}{3}}}</math> | ||
| + | |style="text-align:right;"|ואתה הנחת היות המספר ראשון ג' דברי‫'<br> | ||
| + | א"כ תכה ג' בכ"ח וד' מט' ועולה פ"ה ושליש וכך יבא להיות המספר ראשון | ||
| + | |- | ||
| + | | | ||
| + | :You assumed the second is 4 things, so multiply 4 by 28 and 4 [parts] of 9; the result is 113 and 7 [parts] of 9 and this is the second number. | ||
| + | :<math>\scriptstyle{\color{blue}{b=4x=4\sdot\left(28+\frac{4}{9}\right)=113+\frac{7}{9}}}</math> | ||
| + | |style="text-align:right;"|והשני הנחת היותו ד' דברי‫'<br> | ||
| + | א"כ תכה ד' בכ"ח וד' מט' ועולה קי"ג וז' מט' וכך יבא להיות המספר השני | ||
| + | |- | ||
| + | |Know that this equation can be returned to the first chapter by cubing this way: | ||
| + | |style="text-align:right;"|ודע כי זאת ההשוא' אפש' להשיבה אל הפרק הראשו' בהכות עצמו באופן מעו' בזה האופן | ||
| + | |- | ||
| + | |Multiplying a cube root of a thing by a cube root of a thing generates a cube root of a square. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\sqrt[3]{x}\sdot\sqrt[3]{x}=\sqrt[3]{x^2}}}</math> | ||
| + | |style="text-align:right;"|בהכות שרש מעו' מדבר בשרש מעו' מדבר ועושה שרש מעו' מצינסו | ||
| + | |- | ||
| + | |A cube root of a square by a cube root of a thing generates a cube root of a cube, which is the thing. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\sqrt[3]{x^2}\sdot\sqrt[3]{x}=\sqrt[3]{x^3}=x}}</math> | ||
| + | |style="text-align:right;"|ושרש מעו' מצינסו בשרש מעו' מדבר יעשה שרש מעו' ממעו' שזהו הדבר | ||
| + | |- | ||
| + | |Restore the things this way. | ||
| + | |style="text-align:right;"|וכן תשיב הדברי‫' | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== Chapter 49 ==== | ||
| + | |||
| + | |style="width:45%;text-align:right;"|<big>פרק מ"ט</big> | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|עוד באופן אחר | ||
| + | |- | ||
| + | |{{#annot:ax²=³√c|701|rGD5}}When squares are equal to a cube root of the numbers: | ||
| + | :<math>\scriptstyle ax^2=\sqrt[3]{c}</math> | ||
| + | |style="text-align:right;"|כאשר הצינסי יהיו שוים אל שרשי' מעו' ממספרי‫'{{#annotend:rGD5}} | ||
| + | |- | ||
| + | |The number of the squares should be cubed. | ||
| + | |style="text-align:right;"|צריך להשיב כמות הצינסי אל מעוק‫' | ||
| + | |- | ||
| + | |Then, the radicand of the cube root is divided by cubed number of the squares. | ||
| + | |style="text-align:right;"|ואח"כ לחלק המספרי' הנקובי' להיות להם שרש מעו' בהבאת כמות הצינסי אל מעו‫' | ||
| + | |- | ||
| + | |The [square] root of a cube root of the result is equal to the thing. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{x=\sqrt[6]{\frac{c}{a^3}}}}</math> | ||
| + | |style="text-align:right;"|ושרש משרש ממעו' והעולה מזה שוה הדבר | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:a/b=⅗;ab=³√729|618|ai8Z}}Find me two numbers such that one is a part of the other as 3 is of 5; | ||
| + | :and the one multiplied by the other yields a cube root of 729. | ||
| + | :I ask: how much will each number be? | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle a:b=3:5\\\scriptstyle a\sdot b=\sqrt[3]{729}\end{cases}</math> | ||
| + | |style="text-align:right;"|והנה המשל תמצא לי ב' מספרי' שיהיה האחד חלק מהאחר כמו ג' מה‫'<br> | ||
| + | ומוכה הראשון בשני יעשה שרש מעו' מתשכ"ט<br> | ||
| + | אשאל כמה יבא להיות כל אחד מהמספרי‫'{{#annotend:ai8Z}} | ||
| + | |- | ||
| + | | | ||
| + | :Suppose the first number is three things [<math>\scriptstyle{\color{blue}{a=3x}}</math>] and the second is five things [<math>\scriptstyle{\color{blue}{b=5x}}</math>] | ||
| + | |style="text-align:right;"|תניח שהמספר ראשון יהיה ג' דברי' והשני יהיה ה' דברי‫' | ||
| + | |- | ||
| + | | | ||
| + | :Now, multiply the first by the second, which is 3 things by 5 [things]; the result is 15 squares that are equal to a cube root of 729. | ||
| + | :<math>\scriptstyle{\color{blue}{3x\sdot5x=15x^2=\sqrt[3]{729}}}</math> | ||
| + | |style="text-align:right;"|עתה תכה הראשון בשני וזהו ג' דברי' בה' ועולה ט"ו צינסי שהם שוים אל שרש מעו' מתשכ"ט | ||
| + | |- | ||
| + | | | ||
| + | :Pursue the above given rule: | ||
| + | |style="text-align:right;"|עתה תרדוף כפי הכלל הנתון למעלה | ||
| + | |- | ||
| + | | | ||
| + | :Cube the number of the squares, which is 15; the result is 3375. | ||
| + | |style="text-align:right;"|תשיב כמות הצינסי שהם ט"ו אל מעו' ועולה ג' אלפי' ושע"ה | ||
| + | |- | ||
| + | | | ||
| + | :Divide the radicand of the cube root, which is 729, by 3375; the result is 27 [parts] of 125. | ||
| + | |style="text-align:right;"|ותחלק המספרי' הנקובי' להיות להם שרש מעו' שזהו תשכ"ט על ג' אלפי' ושע"ה ויבא מזה כ"ז מקכ"ה | ||
| + | |- | ||
| + | | | ||
| + | :The square root of the cube root, or say: the cube root of the square root of 27 [parts] of 125 is equal to the thing. | ||
| + | :<math>\scriptstyle{\color{blue}{x=\sqrt[6]{\frac{729}{15^3}}=\sqrt[6]{\frac{729}{3375}}=\sqrt[6]{\frac{27}{125}}}}</math> | ||
| + | |style="text-align:right;"|ושרש המרו' משרש המעו' או תאמ' שרש המעו' משרש המרוב' מכ"ז מקכ"ה יבא לשוות הדבר | ||
| + | |- | ||
| + | | | ||
| + | :You assumed the first number is 3 things, so multiply 3 by a cube root of a square root of 27 [parts] of 125; the result is a square root of a cube root of [157] plus 58 [parts] of 125, which is a square root of 5 and 2-fifths and so is the first number. | ||
| + | :<math>\scriptstyle{\color{blue}{a=3x=3\sdot\sqrt[6]{\frac{27}{125}}=\sqrt[6]{1{\color{red}{57}}+\frac{58}{125}}=\sqrt{5+\frac{2}{5}}}}</math> | ||
| + | |style="text-align:right;"|ואתה הנחת היות המספר ראשון ג' דברי‫'<br> | ||
| + | א"כ תכה ג' בשרש מעו' משרש מרוב' מכ"ז מקכ"ה ועולה שרש מרו' משרש מעוק' מקכ"ה ונ"ח מקכ"ה<br> | ||
| + | אשר בא להיות שרש מרוב' מה' וב' חמישיו' וכך יבא לשוות המספ' להיות המספר ראשון | ||
| + | |- | ||
| + | | | ||
| + | :You assumed the second is 5 things, so multiply 5 by a cube root of a square root of 27 [parts] of 125; the result is a square root of a cube root of 3375, which is a square root of 15 and so is the second number. | ||
| + | :<math>\scriptstyle{\color{blue}{b=5x=5\sdot\sqrt[6]{\frac{27}{125}}=\sqrt[6]{3375}=\sqrt{15}}}</math> | ||
| + | |style="text-align:right;"|והשני הנחת היותו ה' דברי‫'<br> | ||
| + | א"כ תכה ה' בשרש מעו' משרש מרוב' מכ"ז מקכ"ה ועולה שרש מרו' משרש מעו' מג' אלפי' ושע"ה<br> | ||
| + | אשר יבא להיות שרש מרוב' מט"ו וכך יבא המספר השני | ||
| + | |- | ||
| + | |Know that this equation can be returned to chapter 21: | ||
| + | |style="text-align:right;"|ודע כי זאת ההשואה אפש' להשיב' אל הפרק הכ"א | ||
| + | |- | ||
| + | |Since the squares [the are equal] to the cube root of the numbers become cubes [that are equal] to the square root of the numbers. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{ax^2=\sqrt[3]{c}\longrightarrow ax^3=\sqrt{c}}}</math> | ||
| + | |style="text-align:right;"|מפני כי כך יבאו להיות הצינסי אל שרש מעו' ממספרי' כמו המעו' אל שרש מרוב' ממספרי‫' | ||
| + | |- | ||
| + | |Because, by cubing the squares, they becaome squares of squares of square, or cubes of cubes. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\left(x^2\right)^3=x^2x^2x^2=x^3x^3}}</math> | ||
| + | |style="text-align:right;"|מפני כי ב{{#annot:term|2620,1430|R41D}}השבת ה{{#annotend:R41D}}צינסי בהכא' באופן המעו' יעשו {{#annot:term|689|IHxD}}צינסי מצינסי מצינסי{{#annotend:IHxD}} או מעו' ממעו‫' | ||
| + | |- | ||
| + | |Likewise, by squaring the cubes, they become squares of squares of squares, or cubes of cubes. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\left(x^2\right)^3=x^2x^2x^2=x^3x^3}}</math> | ||
| + | |style="text-align:right;"|וכדומה לזה יעשה מעו' בהכותו כמו מרוב' צינסי מצינסי מצינסי או מעו' ממעו‫' | ||
| + | |- | ||
| + | |Also, a cubed square root of numbers is equal to a squared root of their cube. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\left(\sqrt{x}\right)^3=\sqrt{x^3}}}</math> | ||
| + | |style="text-align:right;"|וכן שרש מרו' ממספרי' מושב באופן מעו' הכאה שוה ממה שעושה שרש אחד שוה בהיותו מעו' מוכה בעצמו באופן מרו‫' | ||
| + | |- | ||
| + | |Therefore, when the squares that are one part of the equation are cubed they become equal to the numbers that are the radicand of the cube root. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{x^2=\sqrt[3]{c}\longrightarrow\left(x^2\right)^3=c}}</math> | ||
| + | |style="text-align:right;"|א"כ בהכות הצינסי אשר הם א' מהחלקי' מההשוא' באופן מעוק' עושה כמו המספרי' הנקובי' להיות להם שרש מעו' היותם עוד מספרי‫' | ||
| + | |- | ||
| + | |So [the equation] is returned to chapter 21: | ||
| + | |style="text-align:right;"|א"כ בהשיבה אל הפרק הכ"א | ||
| + | |- | ||
| + | |That is, a root of a square of a square of a square i.e. a root of a cube of a cube becomes a cube and it is equal to a square root of a number, [whose cube root is cubed]. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{x^2=\sqrt[3]{c}\;\longleftrightarrow\;x^6=c\;\longleftrightarrow\;x^3=\sqrt{c}}}</math> | ||
| + | |style="text-align:right;"|דהיינו אל שרש צינסי מצינסי מצינסי דהיינו שרש מעו' ממעו' יבא להיות מעו' ויהיה שוה אל שרש מרובע מהמספר מושב אל מעו‫' | ||
| + | |- | ||
| + | |Hence, a cube equals a root of a number. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{ax^3=\sqrt{c}}}</math> | ||
| + | |style="text-align:right;"|א"כ מעו' יהיה שוה אל שרש מספר | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== Chapter 50 ==== | ||
| + | |||
| + | |style="width:45%;text-align:right;"|<big>פרק נ‫'</big> | ||
| + | |- | ||
| + | |{{#annot:c=³√ax²|701|zK2y}}When numbers are equal to a cube root of squares: | ||
| + | :<math>\scriptstyle c=\sqrt[3]{ax^2}</math> | ||
| + | |style="text-align:right;"|כאשר המספרי' יהיו שוים אל שרשי' מעו' מצינסי{{#annotend:zK2y}} | ||
| + | |- | ||
| + | |The numbers should be cubed. | ||
| + | |style="text-align:right;"|צריך להשיב המספרי' אל מעו‫' | ||
| + | |- | ||
| + | |Then, the cubed number is divided by the number of the squares that are the radicand of the cube root. | ||
| + | |style="text-align:right;"|ואותה ההשבה תחלק בכמות הצינסי הנקובי' להיות להם שרש מעו‫' | ||
| + | |- | ||
| + | |The square root of the result is equal to the thing. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{x=\sqrt{\frac{c^3}{a}}}}</math> | ||
| + | |style="text-align:right;"|ושרש מרוב' ממה שיבא מזה יבא לשוות הדבר | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:a/b=⅓;³√a³√b=100|618|AP3O}}Find me two numbers such that the one is a part of the second as 1 is of 3; | ||
| + | :and the cube root of the first multiplied by the cube root of the second yields 100. | ||
| + | :I ask: how much will each number be? | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle a:b=1:3\\\scriptstyle\sqrt[3]{a}\sdot\sqrt[3]{b}=100\end{cases}</math> | ||
| + | |style="text-align:right;"|והנה המשל עשה לי זה החשבון תמצא לי ‫<ref>43r</ref>שני מספרי' שיהיה הראשון חלק מהשני כמו שא' הוא מג‫'<br> | ||
| + | ומוכה שרש מעו' מהראשון בשרש מעו' השני יעשה ק‫'<br> | ||
| + | אשאל כמה יבא להיות כל אחד מן המספרי‫'{{#annotend:AP3O}} | ||
| + | |- | ||
| + | | | ||
| + | :This is its procedure: | ||
| + | |style="text-align:right;"|זאת היא פעולתו | ||
| + | |- | ||
| + | | | ||
| + | :Suppose the first number is a thing [<math>\scriptstyle{\color{blue}{a=x}}</math>] and the second is three things [<math>\scriptstyle{\color{blue}{b=3x}}</math>]. | ||
| + | |style="text-align:right;"|<big>תניח</big> שהמספר ראשון יהיה דבר אחד והשני ג' דברי‫' | ||
| + | |- | ||
| + | | | ||
| + | :Now, multiply the cube root of the first by the cube root of the second, which is a cube root of a thing by a cube root of 3 things; the result is a cube root of 3 squares and they are equal to 100. | ||
| + | :<math>\scriptstyle{\color{blue}{\sqrt[3]{x}\sdot\sqrt[3]{3x}=\sqrt[3]{3x^2}=100}}</math> | ||
| + | |style="text-align:right;"|עתה תכה שרש המעו' מהראשון עם השרש מעו' מהשני שזהו שרש מעו' דבר אחד בשרש מעו' ג' דברי' ועולה שרש מעו' מג' צינסי והם שוים אל ק‫' | ||
| + | |- | ||
| + | | | ||
| + | :Now, pursue the above mentioned rule: | ||
| + | |style="text-align:right;"|<big>עתה</big> תרדוף כפי הכלל האמור למעלה | ||
| + | |- | ||
| + | | | ||
| + | :Cube a hundred that is the numbers; you get 1000000. | ||
| + | |style="text-align:right;"|תשיב מאה שהם המספרי' אל מעו' ויהיה לך אלף אלפים | ||
| + | |- | ||
| + | | | ||
| + | :Divide it by the number of the squares that are the radicand of the cube root, which is 3; the result is 333333 and one-third. | ||
| + | |style="text-align:right;"|תחלקם בכמות הצינסי הנקובי' להיות להם שרש מעו' שזהו ג' ויבא מזה של"ג אלפי' ושל"ג ושליש | ||
| + | |- | ||
| + | | | ||
| + | :The square root of this is equal to the thing and you assumed the first number is a thing, so the first number is a root of 333333 and one-third. | ||
| + | :<math>\scriptstyle{\color{blue}{a=x=\sqrt{\frac{100^3}{3}}=\sqrt{\frac{1000000}{3}}=\sqrt{333333+\frac{1}{3}}}}</math> | ||
| + | |style="text-align:right;"|ושרש המרו' מזה יבא לשוות הדבר <big>ואתה</big> הנחת היות המספר ראשון דבר אחד א"כ המספר <sup>ראשו'</sup> יבא להיות שרש משל"ג אלפי' ושל"ג ושליש | ||
| + | |- | ||
| + | | | ||
| + | :You assumed the second is 3 things, so multiply 3 by 333333 and one-third; the result is a root of 3000000 and so is the second number. | ||
| + | :<math>\scriptstyle{\color{blue}{b=3x=3\sdot\sqrt{333333+\frac{1}{3}}=\sqrt{3000000}}}</math> | ||
| + | |style="text-align:right;"|והשני הנחת היותו ג' דברי‫'<br> | ||
| + | א"כ תכה ג' בשל"ג אלפי' ושל"ג ושליש ועולה שרש מג' אלפי אלפי' וככה יבא להיות המספר השני | ||
| + | |- | ||
| + | |Know that this equation can be returned to the second chapter, by converting the number to a cube. | ||
| + | |style="text-align:right;"|<big>ודע</big> שזאת ההשואה אפשר להשיבה אל הפרק השני בהשיב המספר אל מעו‫' | ||
| + | |- | ||
| + | |"A cube [root] of a number is equal to a cube root of squares" is equal to your saying "the number is equal to squares". | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\sqrt[3]{c}=\sqrt[3]{ax^2}\;\longleftrightarrow\;c^3=ax^2}}</math> | ||
| + | |style="text-align:right;"|יהיה מעו' מספר שוה אל שרש מעו' מצינסו ושוה לאמרך המספר שוה לצינסי | ||
| + | |} | ||
| + | {| | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== Chapter 51 ==== | ||
| + | |||
| + | |style="width:45%;text-align:right;"|<big>פרק נ"א</big> | ||
| + | |- | ||
| + | | | ||
| + | |style="text-align:right;"|עוד רצוני לשומך באופן אחר | ||
| + | |- | ||
| + | |{{#annot:ax³=³√c|718|eOI5}}It is when cubes are equal to a cube root of the numbers: | ||
| + | :<math>\scriptstyle ax^3=\sqrt[3]{c}</math> | ||
| + | |style="text-align:right;"|וזהו כאשר המעוקבי' יהיו שוים אל שרש מעו' ממספרי‫'{{#annotend:eOI5}} | ||
| + | |- | ||
| + | |The number of the cubes should be cubed. | ||
| + | |style="text-align:right;"|צריך להשיב כמות <sup>המעו'</sup> המספרי' אל מעו‫' | ||
| + | |- | ||
| + | |Then, the radicand of the cube root is divided by the cubed number of the cubes. | ||
| + | |style="text-align:right;"|ולחלק כמות המספרי' הנקובי' להיות להם שרש מעו' בהשבת כמות המעו' אל מעו‫' | ||
| + | |- | ||
| + | |The cube root of the result is equal to the thing and its cube root is a [cube] root of its cube root. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{x=\sqrt[9]{\frac{c}{a^3}}}}</math> | ||
| + | |style="text-align:right;"|ושרש המעו' ממה שיבא מזה ישוה הדבר ושרשו המעוק' הוא {{#annot:term|2634|v7op}}שרש משרשו המעו‫'{{#annotend:v7op}} | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:a/b=¼;aba=³√216|618|nkyB}}Find me two numbers such that one is a part of the other as 1 is of 4; | ||
| + | :and the one multiplied by the other, and this product is multiplied by the first, yields a cube root of 216. | ||
| + | :I ask: how much will each number be? | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle a:b=1:4\\\scriptstyle\left(a\sdot b\right)\sdot a=\sqrt[3]{216}\end{cases}</math> | ||
| + | |style="text-align:right;"|<big>והנה</big> המשל תמצא לי שני מספרי' שיהיה אחד מהם חלק מהאחר כמו שא' הוא מד‫'<br> | ||
| + | ומוכה הראשון בשני וההכאה ההיא תוכה בראשון יעשה שרש מעו' מרי"ו<br> | ||
| + | אשאל כמה יבא להיות כל אחד מהמספרי‫'{{#annotend:nkyB}} | ||
| + | |- | ||
| + | | | ||
| + | :This is its procedure: | ||
| + | |style="text-align:right;"|זו היא פעלתו | ||
| + | |- | ||
| + | | | ||
| + | :Suppose the first number is a thing [<math>\scriptstyle{\color{blue}{a=x}}</math>] and the other is four things [<math>\scriptstyle{\color{blue}{b=4x}}</math>] | ||
| + | |style="text-align:right;"|תניח שהמספר ראשון יהיה דבר אחד והאחר יהיה ד' דברי‫' | ||
| + | |- | ||
| + | | | ||
| + | :Multiply the first by the second, which is a thing by 4 things; the result is 4 squares. | ||
| + | :<math>\scriptstyle{\color{blue}{x\sdot4x=4x^2}}</math> | ||
| + | |style="text-align:right;"|עתה תכה הראשון בשני שזהו דבר אחד בד' דברי' עולה ד' צינסי | ||
| + | |- | ||
| + | | | ||
| + | :Multiply this product, which is 4 squares, by the first number, which is a thing; the result is 4 cubes that are equal to a cube root of 216. | ||
| + | :<math>\scriptstyle{\color{blue}{4x^2\sdot x=4x^3=\sqrt[3]{216}}}</math> | ||
| + | |style="text-align:right;"|וזאת ההכאה שהיא ד' צינסי תכה במספר ראשו' שהוא דבר אחד עולה ד' מעו' שהם שוים אל שרש מעו' מרי"ו | ||
| + | |- | ||
| + | | | ||
| + | :Now, pursue the above mentioned rule | ||
| + | |style="text-align:right;"|<big>עתה</big> תרדוף כפי הכלל האמור למעלה | ||
| + | |- | ||
| + | | | ||
| + | :You should cube the number of the cubes, which is 4; you get 64. | ||
| + | |style="text-align:right;"|הנך צריך להשיב כמות המעו' אל מעו' וזהו ד' ויהיה לך <s>י"ו</s> ס"ד | ||
| + | |- | ||
| + | | | ||
| + | :Divide the radicand of the cube root, which is 216, by 64; the result is 3 and 3 [parts] of 8. | ||
| + | |style="text-align:right;"|ותחלק כמות המספרי' הנקובי' להיות להם שרש מעו' והם רי"ו על ס"ד ו{{#annot:term|875,1566|A46L}}יבא מזה{{#annotend:A46L}} ג' וג' מח‫' | ||
| + | |- | ||
| + | | | ||
| + | :The cube root of the cube root of 3 and 3 [parts] of 8 is the thing and so is the first number you assumed is a thing. | ||
| + | :<math>\scriptstyle{\color{blue}{a=x=\sqrt[9]{\frac{216}{4^3}}=\sqrt[9]{\frac{216}{64}}=\sqrt[9]{3+\frac{3}{8}}}}</math> | ||
| + | |style="text-align:right;"|ו{{#annot:term|2634|2lKu}}שרש מעו' משרש מעו' מ{{#annotend:2lKu}}ג' וג' מח' יבא להיות הדבר וככה יבא להיות המספר ראשון אשר הנחת היותו דבר אחד | ||
| + | |- | ||
| + | | | ||
| + | :You assumed the second is a thing, so, multiply 4 by a cube root of a cube root of 3 and 3 [parts] of 8. | ||
| + | |style="text-align:right;"|והשני הנחת היותו ד' דברי' א"כ תכה ד' בשרש מעו' משרש מעו' מג' וג' מח‫' | ||
| + | |- | ||
| + | | | ||
| + | :But, remember that you have to convert [the 4] into a cube root of a cube root; you get a cube root of a cube root of 262144. | ||
| + | |style="text-align:right;"|ותזכור כי הנך צריך להשיב אל {{#annot:term|2634|tWs7}}שרש מעו' משרש מעו'{{#annotend:tWs7}} ויהיה לך שרש מעו' משרש מעו' מרס"ב אלפי' וקמ"ד | ||
| + | |- | ||
| + | | | ||
| + | :Multiply it by 3 and 3 [parts] of 8; the result is a cube root of a cube root of 884736 and so is the second number. | ||
| + | :<math>\scriptstyle{\color{blue}{b=4x=4\sdot\sqrt[9]{3+\frac{3}{8}}=\sqrt[9]{262144\sdot\left(3+\frac{3}{8}\right)}=\sqrt[9]{884736}}}</math> | ||
| + | |style="text-align:right;"|וזה תכה בג' וג' מח' ועולה שרש מעו' משרש מעו' מתתפ"ד אלפי' ותשל"ו וככה יבא להיות המספ' השני‫<ref>Jerusalem end.</ref> | ||
| + | |- | ||
| + | |Over and done | ||
| + | |style="text-align:right;"|תם ונשלם | ||
| + | |- | ||
| + | |Praise to the Creator of the Universe | ||
| + | |style="text-align:right;"|שבח לבורא עולם | ||
| + | |} | ||
| + | |||
| + | == Notes == | ||
| + | |||
| + | <div class="mw-collapsible mw-collapsed"><div class="mw-collapsible-content"> | ||
| + | <references /> | ||
| + | </div></div> | ||
| + | |||
| + | == Appendix: Bibliography == | ||
| + | '''''Aliabraa Argibra''''' / by Maestro Dardi (Pisa, 14th century)<br> | ||
| + | – Hebrew translation –<br> | ||
| + | by Mordecai (Angelo) Finzi (Mantua, d. 1475)<br> | ||
| + | '''''Jīblī al-Mūqabāla'''<br> | ||
'''Manuscripts:'''<br> | '''Manuscripts:'''<br> | ||
| − | + | *Jerusalem, The National Library of Israel Ms. Heb. 8°3915 (IMHM: B 546 (8°3915)), ff. 1r-43r (Mantova, 1473-1975; autograph) | |
| − | #Paris, Bibliothèque Nationale de France heb. 1029/5 (IMHM: f 15721), ff. 194r-234r (15th-16th century) | + | ::[[https://www.nli.org.il/he/discover/manuscripts/hebrew-manuscripts/viewerpage?vid=MANUSCRIPTS&docid=PNX_MANUSCRIPTS990000427200205171-1#$FL71441025 Jerusalem 8°3915]] |
| − | + | *Paris, Bibliothèque Nationale de France heb. 1029/5 (IMHM: f 15721), ff. 194r-234r (15th-16th century) | |
| + | ::[[https://gallica.bnf.fr/ark:/12148/btv1b9064672r/f195.item.r=Hébreu.langEN.zoom Paris1029]] | ||
| + | *Paris, Bibliothèque Nationale de France heb. 1033/2 (IMHM: f 15026), ff. 91r-150v (16th century) | ||
| + | ::[[https://gallica.bnf.fr/ark:/12148/btv1b105446161/f206.image.r=1033 Paris1033]] | ||
| + | |||
| + | <span style=color:blue>The transcript is based mainly on manuscript Jerusalem 8°3915</span> | ||
'''<u>Critical Edition (of the first section)</u>''': | '''<u>Critical Edition (of the first section)</u>''': | ||
Latest revision as of 17:03, 9 October 2022
Contents
[hide]- 1 Table of Contents
- 2 Prologue
- 3 Roots
- 3.1 Multiplication of Roots
- 3.1.1 Multiplication of a Root by a Root
- 3.1.2 Multiplication of a Root of a Number by a Number
- 3.1.3 Multiplication of a Root of a Number by a Number and a Root of a Number
- 3.1.4 Multiplication of a Number minus a Root by a Root
- 3.1.5 Multiplication of a Number and a Root by a Number and a Root - when the numbers are identical and the roots are identical
- 3.1.6 Multiplication of a Number and a Root by a Number and a Root
- 3.1.7 Multiplication of a Number minus a Root by a Number minus a Root
- 3.1.8 Multiplication of a Number minus a Root by a Number minus a Root - when the numbers are identical and the roots are identical
- 3.1.9 Multiplication of a Number and a Root by a Number minus a Root - when the numbers are identical and the roots are identical
- 3.1.10 Multiplication of a Number and a Root by a Number minus a Root
- 3.1.11 Multiplication of a Root by a Root minus a Number
- 3.1.12 Multiplication of a Root minus a Number by a Root minus a Number
- 3.1.13 Multiplication of a Root minus a Number by a Root minus a Number - when the numbers are identical [and the roots are identical]
- 3.1.14 Multiplication of a Root minus a Number by a Root and a Number
- 3.1.15 Multiplication of a Root and a Number by a Root minus a Number - when the numbers are identical and the roots are identical
- 3.1.16 Multiplication of a Root by a Root and a Root
- 3.1.17 Multiplication of a Root by a Root minus a Root
- 3.1.18 Multiplication of Two Roots by Two other Roots
- 3.1.19 Multiplication of Two Roots by Two other Roots - when the first roots of both parts are identical and the second roots of both parts are identical
- 3.1.20 Multiplication of a Root and a Root by a Root minus a Root
- 3.1.21 Multiplication of a Root and a Root by a Root minus a Root - when the first roots of both parts are identical and the second roots of both parts are identical
- 3.1.22 Multiplication of a Root minus a Root by a Root minus a Root
- 3.1.23 Multiplication of a Root minus a Root by a Root minus a Root - when the first roots of both parts are identical and the second roots of both parts are identical
- 3.1.24 Multiplication of a Number by a Root
- 3.1.25 Multiplication of a Square Root by a Cubic Root
- 3.1.26 Multiplication of a Cube Root by a Root of a Root
- 3.1.27 Multiplication of a Number and a Root of a Number and a Root by a Number and a Root of a Number and a Root
- 3.2 Addition and Subtraction of Roots
- 3.2.1 Addition of a Root with a Root
- 3.2.2 Subtraction of a Root from a Root
- 3.2.3 Addition of a Number and a Root with a Number and a Root
- 3.2.4 Addition of a Number and a Root with a Root minus a Number
- 3.2.5 Addition of a Number minus a Root with a Number minus a Root
- 3.2.6 Subtraction of a Number minus a Root from a Number
- 3.2.7 Subtraction of a Number and a Root from a Number
- 3.2.8 Subtraction of a Number minus a Root from a Number
- 3.2.9 Subtraction of a Number minus a Root from a Number minus a Root
- 3.2.10 Addition of Numerous Roots
- 3.3 Division of Roots
- 3.3.1 Division of a Number by a Root
- 3.3.2 Division of a Number by a Root and a Number
- 3.3.3 Division of a Number and a Root by a Number
- 3.3.4 Division of a Number by a Number minus a Root
- 3.3.5 Division of a Number and a Root by a Number and a Root
- 3.3.6 Division of a Number by Three Roots
- 3.3.7 Division of a Number by Four Roots
- 3.1 Multiplication of Roots
- 4 Algebra
- 4.1 The Six Canonical Equations
- 4.2 Complex Equations
- 4.2.1 Chapter Seven
- 4.2.2 Chapter 8
- 4.2.3 Chapter Nine
- 4.2.4 Chapter Ten
- 4.2.5 Chapter 11
- 4.2.6 Chapter 12
- 4.2.7 Chapter 13
- 4.2.8 Chapter 14
- 4.2.9 Chapter 15
- 4.2.10 Chapter 16
- 4.2.11 Chapter 17
- 4.2.12 Chapter 18
- 4.2.13 Chapter 19
- 4.2.14 Chapter 20
- 4.2.15 Chapter 21
- 4.2.16 Chapter 22
- 4.2.17 Chapter 23
- 4.2.18 Chapter 24
- 4.2.19 Chapter 25
- 4.2.20 Chapter 26
- 4.2.21 Chapter 27
- 4.2.22 Chapter 28
- 4.2.23 Chapter 29
- 4.2.24 Chapter 30
- 4.2.25 Chapter 31
- 4.2.26 Chapter 32
- 4.2.27 Chapter 33
- 4.2.28 Chapter 34
- 4.2.29 Chapter 35
- 4.2.30 Chapter 36
- 4.2.31 Chapter 37
- 4.2.32 Chapter 38
- 4.2.33 Chapter 39
- 4.2.34 Chapter 40
- 4.2.35 Chapter 41
- 4.2.36 Chapter 42
- 4.2.37 Chapter 43
- 4.2.38 Chapter 44
- 4.2.39 Chapter 45
- 4.2.40 Chapter 46
- 4.2.41 Chapter 47
- 4.2.42 Chapter 48
- 4.2.43 Chapter 49
- 4.2.44 Chapter 50
- 4.2.45 Chapter 51
- 5 Notes
- 6 Appendix: Bibliography
| These are the signs of the six abbreviated chapters of the book Jīblī al-Mūqabāla, | [1]אלו הם הסימנים מהששה פרקים מקוצרים מספר גבלי אלמוקבאלא |
| and of those written and explained by Master Dardi from Pisa, | ומאשר האומן דארדי מפיסא כתב ופירש עליהם |
| which are 194 in number. | אשר הם במספר קצ"ד |
| The names of the quantities that will be spoken of are five, which are: | שמות הכמויות אשר ידובר בהם הם חמשה והם |
|
מספר או דראמא |
|
דבר או שורש |
|
צינסו |
|
ומעוקב |
|
וצינסו מצינסו |
| [Their abbreviations]: | |
|
ובמקום המספר או הדראמא אנו נשים מ' או דר' |
|
ובמקום הדבר או שרש אנחנו נשים ד' או ש' |
|
ובמקום הצינסו צ' |
|
ובמקום המעוקב מעו' |
|
ובעד הצינסו דצינסו צ' מצ' |
| As is seen in the categories from here on. | כפי אשר תראה בחלוקות מכאן ולהבא |
| First the thing is defined. | וראשונה נשים הדבר |
Table of Contents |
|
|
פרק א' דבר שוה למספר |
|
פרק ב' צינסו שוה למספר |
|
פרק ג' צינסו שוה לדבר |
|
פרק ד' צינסו ודבר למספר |
|
פרק ה' צינסו ומספר לדבר |
|
פרק ו' דבר ומספר לצינסו |
|
פרק ז' מעוקב שוה למספר |
|
פרק ח' מעוקב לדבר |
|
פרק ט' מעוקב לצינסו |
|
פרק י' מעו' לצינסו מצינסו |
|
פרק י"א צינסו דצינסו למספר |
|
פרק י"ב צינסו דצינסו לדבר |
|
פרק י"ג צינסו דצינסו לצינסו |
|
פרק י"ד מעו' וצינסו לדבר |
|
פרק ט"ו מעו' ודבר לצינסו |
|
פרק י"ו צינסו ודבר למעו' |
|
פרק י"ז מספר לשרש הדבר |
|
פרק י"ח דבר לשרש המספר |
|
פרק י"ט צינ' לשרש המספר |
|
פרק כ' מספר לשרש צינ' |
|
פרק כ"א מעו' לשרש מספר |
|
פרק כ"ב מספר לשרש מעו' |
|
פרק כ"ג צי' מצי' לשרש מספר |
|
פרק כ"ד מס' לשרש צינסו מצי' |
|
פרק כ"ה דבר לשרש דבר |
|
פרק כ"ו צינסו לשרש דבר |
|
פרק כ"ז דבר לשרש מעוק' |
|
פרק כ"ח דבר לשרש צינ' מצי' |
|
פרק כ"ט צינסו לשרש צינסו |
|
פרק ל' צינסו לשרש מעו' |
|
פרק ל"א מעוק' לשרש צינסו |
|
פרק ל"ב צי' מצי' לשרש צינסו |
|
פרק ל"ג מעוק' לשרש מעוקב |
|
פרק ל"ד מעו' לשרש צינ' מצי' |
|
פרק ל"ה צי' מצי' לשרש צי' מצי' |
|
פרק ל"ו דבר למספ' ולשרש מספ' |
|
פרק ל"ז מס' לדבר ולשרש דבר |
|
פרק ל"ח צי' למספר ולשרש מספ' |
|
פרק ל"ט מס' לצינ' ולשרש צינ' |
|
פרק מ' מעו' למספ' ולשרש מס' |
|
פרק מ"א מס' למעו' ולשרש מעו' |
|
פרק מ"ב צי' מצי' למס' ולשרש מס' |
|
פרק מ"ג מספ' לצי' מצי' ולש' צי' מצי' |
|
פרק מ"ד צי' מצי' וצינ' למספר |
|
פרק מ"ה צי' לצי' מצי' ולמספר |
|
פרק מ"ו צי' מצי' לצי' ולמספר |
|
[2]פרק מ"ז צינסו לצינ' מצי' ולמספר |
|
פרק מ"ח מספ' לשרש מעו' מדבר |
|
פרק מ"ט צינסו לשרש מעו' ממספ' |
|
פרק נ' מספר לשרש מעו' מצי' |
|
פרק נ"א מעו' לשרש מעו' ממס' |
|
פרק נ"ב מס' לשרש מעו' ממס' |
|
פרק נ"ג צינ' מצי' לשרש מעו' ממס' |
|
פרק נ"ד מס' לשרש מעו' מצי' מצי' |
|
פרק נ"ה דבר לשרש מעו' מדבר |
|
פרק נ"ו דבר לשרש מעו' מצי' |
|
פרק נ"ז מעו' לשרש מעו' מדבר |
|
פרק נ"ח דב' לשרש מעו' מצי' מצי' |
|
פרק נ"ט צי' לשרש מעו' מצי' |
|
פרק ס' צי' לשרש מעו' ממעו' |
|
פרק ס"א צי' לשרש מעו' צי' מצי' |
|
פרק ס"ב מעו' לשרש מעו' ממעו' |
|
פרק ס"ג צי' מצי' לשרש מעו' ממעו' |
|
פרק ס"ד צי' מצי' לשרש מעו' צי' מצי' |
|
פרק ס"ה דבר למס' ולשרש מספר |
|
פרק ס"ו צי' למס' ולשרש מעו' ממס' |
|
פרק ס"ז מעו' למס' ולש' מעו' ממס' |
|
פרק ס"ח צי' מצי' למס' ולש' מעו' ממס' |
|
פרק ס"ט צי' מצי' ומעו' לצינ' |
|
פרק ע' צי' מצי' וצינ' למעוקב |
|
פרק ע"א צי' מצי' לצי' ולמעו' |
|
פרק ע"ב צי' ודבר לשרש מספ' |
|
פרק ע"ג צינ' ושרש מס' לדבר |
|
פרק ע"ד דבר ושרש מספ' לצי' |
|
פרק ע"ה צי' מצי' וצי' לשרש מספ' |
|
פרק ע"ו צי' מצי' ושרש מס' לצי' |
|
פרק ע"ז צי' מצי' לצי' וש' מספר |
|
פרק ע"ח דבר ושרש צי' למס' |
|
פרק ע"ט מס' ושרש צי' לדבר |
|
פרק פ' דבר ומס' לשרש צינ' |
|
פרק פ"א צי' ושרש צי' לדבר |
|
פרק פ"ב דבר ושרש צי' לצי' |
|
פרק פ"ג צי' ודבר לשרש צי' |
|
פרק פ"ד צי' ושרש מעו' לדבר |
|
פרק פ"ה דבר ושרש מעו' לצי' |
|
פרק פ"ו דבר וצי' לשרש מעו' |
|
פרק פ"ז מס' ושרש דבר לדבר |
|
פרק פ"ח מספ' ושרש צי' לצי' |
|
פרק פ"ט מספר ודבר לש' דבר |
|
פרק צ' מס' וצי' לשרש צינ' |
|
פרק צ"א מספ' ושרש מעו' למעו' |
|
פרק צ"ב מספ' ומעו' לשרש מעו' |
|
פרק צ"ג מס' וש' צי' מצי' לצי' |
|
פרק צ"ד מס' וצי' מצי' לשרש צי' מצי' |
|
פרק צ"ה צי' ודבר לשרש מעו' ממס' |
|
פרק צ"ו צי' וש' מעו' ממס' לדבר |
|
פרק צ"ז דבר וש' מעו' ממס' לצי' |
|
פרק צ"ח צי' מצי' וצי' לש' מעו' ממס' |
|
פרק צ"ט צי' מצי' וש' מעו' ממס' לצי' |
|
פרק ק' צי' מצי' לצי' ולש' מעו' ממס' |
|
פרק ק"א דבר ומס' לש' מספר |
|
פרק ק"ב דבר ושרש מספ' למס' |
|
פרק ק"ג צי' ומס' לשרש מספ' |
|
פרק ק"ד צי' ושרש מס' למספר |
|
פרק ק"ה מעו' ומס' לש' מספר |
|
פרק ק"ו מעו' ושרש מס' למס' |
|
[3]פרק ק"ז צי' מצי' ומס' לש' מספר |
|
פרק ק"ח צי' מצי' ושרש מס' למס' |
|
פרק ק"ט דבר וש' דבר לש' מספר |
|
פרק קי' דבר וש' מס' לש' דבר |
|
פרק קי"א שרש מס' וש' דבר לדבר' |
|
פרק קי"ב צי' וש' צי' לשרש מספר |
|
פרק קי"ג צי' וש' מס' לשרש צינ' |
|
פרק קי"ד שרש צי' וש' מספ' לצי' |
|
פרק קט"ו מעו' וש' מעו' לש' מס' |
|
פרק קי"ו מעו' וש' מספ' לש' מעו' |
|
פרק קי"ז שרש מעו' ושרש מס' למעו' |
|
פרק קי"ח צי' מצי' וש' צי' מצי' לש' מס' |
|
פרק קי"ט צי' מצי' וש' מס' לש' צי' מצי' |
|
פרק ק"כ ש' צי' מצי' וש' מס' לצי' מצי' |
|
פרק קכ"א דבר וש' דבר לש' מעו' ממס' |
|
פרק קכ"ב דבר וש' מעו' ממס' לש' דב' |
|
פרק קכ"ג ש' צי' וש' מעו' ממס' לדבר |
|
פרק קכ"ד צי' וש' צי' לש' מעו' ממס' |
|
פרק קכ"ה צי' וש' מעו' ממס' לש' צי' |
|
פרק קכ"ו ש' צי' וש' מעו' ממס' לצי' |
|
פרק קכ"ז מעו' וש' מעו' לש' מעו' ממס' |
|
פרק קכ"ח מעו' וש' מעו' ממס' למעו' |
|
פרק קכ"ט ש' מעו' וש' מעו' ממס' למעו' |
|
פרק ק"ל צי' מצי' וש' צי' מצי' לש' מעו' מס' |
|
פרק קל"א צי' מצי' וש' מעו' מס' לש' צי' מצי' |
|
פרק קל"ב ש' צי' מצי' וש' מעו' מס' לצי' מצי' |
|
פרק קל"ג צי' ודבר לש' צי' מצינסו |
|
פרק קל"ד דבר וש' מצי' דצי' לדבר |
|
פרק קל"ה דבר וש' צי' מצי' לצי' |
|
פרק קל"ו דבר ומס' לש' מעו' מס' |
|
פרק קל"ז דבר וש' מעו' מס' למס' |
|
פרק קל"ח דבר ומס' לש' מעו' מס' |
|
פרק קל"ט צי' וש' מעו' מס' למס' |
|
פרק ק"מ מעו' ומס' לש' מעו' מס' |
|
פרק קמ"א מעו' וש' מעו' מס' למס' |
|
פרק קמ"ב צי' דצי' ומס' לש' מעו' מס' |
|
פרק קמ"ג צי' מצי' וש' מעו' מס' למס' |
|
פרק קמ"ד ש' מצי' ודבר לש' מספר |
|
פרק קמ"ה ש' צי' וש' מספ' לדבר |
|
פרק קמ"ו דבר וש' מס' לש' צי' |
|
פרק קמ"ז ש' צי' ודבר לש' מעו' מס' |
|
פרק קמ"ח ש' צי' וש' מעו' מס' לדבר |
|
פרק קמ"ט דבר וש' מעו' מס' לש' צי' |
|
פרק ק"ן צי' וש' צי' מצי' למספר |
|
פרק קנ"א מס' וש' צינ' מצי' לצינסו |
|
פרק קנ"ב צי' ומס' לש' צי' מצי' |
|
פרק קנ"ג צי' וש' צי' מצי' לש' מספ' |
|
פרק קנ"ד ש' צי' מצי' וש' מס' לצי' |
|
פרק קנ"ה צי' וש' מס' לש' צי' מצי' |
|
פרק קנ"ו צי' וש' צי' מצי' לש' מעו' מס' |
|
פרק קנ"ז ש' צי' מצי' וש' מעו' מס' לצי' |
|
פרק קנ"ח צי' וש' מעו' מס' לש' צי' מצי' |
|
פרק קנ"ט ש' מעו' דבר למספר |
|
פרק ק"ס ש' מעו' צי' למס' |
|
פרק קס"א ש' מעו' מעו' למספר |
|
פרק קס"ב ש' מעו' צי' מצי' למס' |
|
פרק קס"ג ש' מעו' דבר לש' מס' |
|
פרק קס"ד ש' מעו' צי' לש' מספ' |
|
פרק קס"ה ש' מעו' ממעו' לש' מס' |
|
פרק קס"ו ש' מעו' צי' מצי' לש' מס' |
|
[4]פרק קס"ז שרש דבר לש' מעו' מס' |
|
פרק קס"ח שרש צי' לש' מעו' מספ' |
|
פרק קס"ט שרש מעו' לש' מעו' מס' |
|
פרק ק"ע ש' צי' מצי' לש' מעו' מספ' |
|
פרק קע"א ש' דבר לש' מעו' דבר |
|
פרק קע"ב ש' צי' לש' מעו' צינסו |
|
פרק קע"ג ש' מעו' לש' מעו' ממעו' |
|
פרק קע"ד ש' צי' מצי' לש' מעו' צי' מצי' |
|
פרק קע"ה ש' דבר לש' מעו' צי' |
|
פרק קע"ו שרש דבר לש' מעו' המעו' |
|
פרק קע"ז ש' דבר לש' מעו' צי' מצי' |
|
פרק קע"ח ש' צי' לש' מעו' מדבר |
|
פרק קע"ט ש' מעו' לש' מעו' מדבר |
|
פרק ק"פ ש' צי' מצי' לש' מעו' מצי' |
|
פרק קפ"א ש' מעו' לש' מעו' צי' מצי' |
|
פרק קפ"ב ש' צי' מצי' לש' מעו' המעו' |
|
פרק קפ"ג צי' מצי' למס' ולש' מס' |
|
פרק קפ"ד צי' ומס' וש' מס' לדבר |
|
פרק קפ"ה דבר ומס' וש' מס' לצי' |
|
פרק קפ"ו צי' ודבר למס' ולש' מעו' מס' |
|
פרק קפ"ז צי' ומס' וש' מעו' מס' לדבר |
|
פרק קפ"ח דבר ומס' וש' מעו' מס' לצי' |
|
פרק קפ"ט צי' מצי' וצי' למס' וש' מס' |
|
פרק ק"ץ צי' מצי' ומס' וש' מס' לצי' |
|
פרק קצ"א צי' ומס' וש' מס' לצי' מצי' |
|
פרק קצ"ב צי' מצי' וצי' למס' וש' מעו' ממס' |
|
פרק קצ"ג צי' מצי' ומס' וש' מעו' מס' לצי' |
|
פרק קצ"ד צי' ומס' וש' מעו' מס' לצי' מצי' |
| The chapters mentioned above are laid out in general rules, as seen further in the booklet, on page 121. | הפרקים הנזכרים למעלה הם סודרו מסדרים כוללים כנראה בהמשך הקנטריס בעלה קכ"א |
| Still further, a few chapters are laid out in non inclusive rules, while these rules are truthful for the rules that are given for the amendments of the aforementioned chapters, and these are: | עוד אחר זה אמשיך כמה פרקים אשר הם מסודרים מסדרים בלתי כוללים עם שהסדרים ההם הם אמתיים למשפטים המגיעים לתיקוניהם של הפרקים הנזכרים והם אלו אשר אכתוב |
|
פרק א' דבר וצי' ומעו' למספ' |
|
פרק ב' דבר וצי' ומעו' וצי' מצי' למס' |
|
פרק ג' דב' וצי' מצי' למס' ומעו' |
|
פרק ד' דבר וצי מצי' למס' ולצי' ולמעו' |
| Then, after these chapters, the rules for extracting square root and cube root of any number are given: | עוד ימשכו אחר אלו הפרקים סדר הוצאת ש' מרובע ושרש מעוקב מכל מספר שיהיה |
|
פרק א' סדר למצא שרש המרובע |
|
פרק ב' הסדר למצא שרש המעוקב |
| Know that after the above mentioned rules, many other calculations are calculated, which are beautiful and subtle. | ודע כי אחרי הסדרים הנזכרים יחשבו חשבונות אחרים רבים שהם יפים ודקים |
| Some of them can be defined with the aforesaid rules, | אבל קצתם יתכן לשומם עם הסדרים הנזכרים |
| and some of them cannot, yet they are nice and delightful. | וקצתם לא יתכן אבל הם יפים ומענגים |
Prologue |
|
| In the name of the Lord, Amen | [5]בשם השם אמן |
| This book was translated from another book, first written on November 9, 1344 (Christian Calendar). | זה הספר נעתק מספר אחר אשר נכתב ראשונה בט' נובימ' אלף שמ"ד לחשבו' הנצרים |
| Then, Jacomo di Ierushali da Litovilana, who lives in Mantua on the road of Unicorno, close to the Church of San Barnaba, began writing it on Saturday, May 3, 1429. | ואחר זה התחילו לכתוב אותו יקומו דירושילי דליטובילאנה הדר בעיר מנטואה במסלת האוניקורנו קרוב לקדש ס' בירנבי ביום שבת ג' מאיו [אלף תכ"ט][6] |
| In which many rules beneficial in calculations are kept, as we can see further in this book. | אשר בו יוחזקו סדרים רבים בעלי תועלת מחשבונות כאשר נוכל לראות בהמשך זה הספר |
| I, Mordecai Finzi, started translating it here, in Mantua, from the Christian language to Hebrew, for the benefit of our people, on Wednesday, November 24, year 5234 from the creation [= 1473 C.E.]. | ואני מרדכי פינצי התחלתי להעתיקו הנה מנטואה מן הנצרי אל העברי לתועלת בני עמנו ביום רביעי כ"ד בנובימ' שנת ה' אלפי' ורל"ד ליצירה |
| I have trusted the Lord, I shall not stumble. | ובה' בטחתי לא אמעד[7] |
Roots |
|
| Hereinafter, I start the necessary rules of multiplication and division of roots, as well as summing roots together, subtracting a root from another root, extracting expressible roots of square and cubic numbers, and many other useful rules by which the calculations of the craftsmen are known. | בכאן אתחיל הסדרי' הראויים בהכאה ובחלוקה לשרשים וג"כ לחבר שרשי' יחד ולהוציא שרש משרש אחר וג"כ למצוא שרשי מספרי מרובע ומעוקב המדוברים וסדרי' אחרי' רבים דקים ומועילים אשר עמהם יוכרו חשבונו' בעלי אומנות |
| First, we start with understanding what are square and cube roots of a number, why they are called roots, I mean what is the essence of the root of a number, whether a square or a cube root, and in what way the roots yield a number. | וראשונה נתחיל להבין מה הוא השרש המרובע וג"כ שרש מעוקב ממספר ולמה נקראים שרשים רצוני מהו עצם שרש המספר בין שיהיה שרש מרובע או שרש מעוקב ובאיזה אופן השרשי' יעשו מספר |
| One should first know the nature of the root - as every number is multiplied by itself, the square root is the product of its multiplication. | וראשונה ראוי לדעת טבע השרש בהיות כי כל מספר מוכה בעצמו הנה הוא שרש מרובע העולה מהכאתו |
| It should be known that every number has a square and cube root either visible or hidden. | וראוי לדעת כי לכל מספר יש לו שרש מרובע |
| But, not every number has a visible square and cube root. | אבל אין לכל מספר שרש |
| The numbers that do not have a visible square nor cube root are called deaf or mute, whether square or cube. | אבל אותם המספרי' אשר לא נמצא להם שרש גלוי לא מרובע ולא מעוקב נקרא חרש או אלם מרובע יהיה או מעוקב |
| Since they cannot be expressed or heard by a visible number. | מפני כי לא ידובר בו ולא יאוזן במספר גלוי |
| They are also called continuous or concealed. | וג"כ יקראו תמידי' או לא גלויים |
| Since the numbers are formed by them, but their expression is hidden. | מפני כי בהם יקוימו המספרי' ונעלם הבטוי בו |
| Know that every number that is multiplied by itself, then the product is multiplied by that same number, which is its root, is a cube. | ודע כי כל מספר שיוכה בעצמו והעולה יוכה במספר בעצמו שהם שרשיו הוא מעוקב |
| Since we have told you the nature of the square root, then of the cube root, and the way each of them by itself is found from the number, for which they are called roots, I wish to give you examples for square roots: | ובהיות שאמרנו לך למעלה טבע שרש המרובע ואחר ג"כ משרש המעוקב ובאיזה אופן כל אחד לבדו יגיע המספר אשר בעד המספר ההוא יקראו שרשים רציתי לשים לך דמיון השרש מרובע |
|
האחד הוא שרש לעצמו מפני כי כשיוכה בעצמו לא יגיע רק עצמו רצוני אחד |
|
ושנים הוא שרש מרובע מארבעה ושלשה מתשעה מפני כי כשיוכה ב' בעצמו יעלה ארבעה וג' בעצמו יעלה תשעה |
| In this manner: any root or number is a root of its product by itself | ובזה האופן כל שרש או מספר הוא שרש מאשר יעלה הכאתו בעצמו |
| Examples for cube roots: | ודמיון שרש מעוקב |
|
האחד הוא שרש מעוקב גם כן מעצמו ר"ל מאחד מפני כי כשיוכה אחד על עצמו ואחר זה העולה שהוא אחד יוכה ג"כ במספר ההוא לא יעלה רק עצמו רצוני אחד |
|
ושנים הוא שרש מעוקב לשמנה ושלשה לכ"ז מפני כי כשיוכה ב' בעצמו יעלה ד' [8]וזה העולה רצוני ד' יוכה במספר האמור הוא שנים יעלה שמנה וכשיוכה שלשה על עצמו יעלה תשעה וזה התשעה יוכה במספר האמור הוא שלשה יעלה כ"ז |
|
ולזה האחד הוא שרש מרובע לאחד ושרש מעוקב לאחד |
|
ושנים הוא שרש מרובע לארבעה ושרש מעוקב לשמנה |
|
וג' הוא שרש מרובע לתשעה ושרש מעוקב לכ"ז |
| In this manner the square numbers and the cube numbers are found, and their roots are understood. | ובזה האופן ימצאו המספרי' המרובעי' והמספרי' המעוקבי' והבנת שרשיהם |
| In this order endlessly. | ועל זה הסדר עד לאין תכלית |
Multiplication of Roots |
|
| After you have seen the nature of the roots and the essence of square and cube numbers, and the extraction of their roots, | אחר אשר ראית טבע השרשי' ועצמות המספרי' המרובעי' והמספרי' המעוקבי' איך יצאו משרשיהם |
| From here on, I wish to show you how to multiply one root by another root, or a number by a root, or a number and a root by a number and a root, or a number minus a root by a number minus a root, and any other manner that can be carried out, as you will see in the following teaching of the aforementioned multiplication. | מכאן ולהבא רצוני להראותך כיצד תכפול שרש אחד בשרש אחר או מספר בשרש או מספר ושרש במספר ושרש או מספר פחות שרש במספר פחות שרש וגם כן בכל אופן אחר אשר יוכל להגיע כפי אשר תראה בלמוד הבא בכפל הנזכר |
Multiplication of a Root by a Root |
|
| First I want to show you how we multiply one root simply by another: | וראשונה רצוני להראותך כיצד נכפול שרש אחד באחר פשוט |
|
נניח שרצית לכפול שרש ארבעה בשרש תשעה |
|
הנה צריך שתכפול המספרי' זה על זה שהם ד' על ט' ועלה ל"ו |
|
ושרש זה הל"ו הוא שרש ושרש הל"ו האלה הוא ששה |
| The proof of this example: | והנה הראיה לדמיון |
|
בהיות כי שרש ד' הוא ב' ושרש ט' הוא ג' כאשר הראית למעלה |
|
הנה כאשר יוכה שרש ד' שהוא ב' בשרש ט' שהוא ג' דהינו ב' פעמי' ג' יעשה ו' שהוא באמת שרש ל"ו |
| I have already taught you previously that every number that is multiplied by itself is the root of that product.
|
בהיות כי כבר למדתיך בעבר שכל מספר אשר יוכה בעצמו הנה הוא שרש מהעולה מן ההכאה |
|
ולכן ששה הוא היטב שרש מהכאתו בעצמו שהוא ל"ו |
| According to this example, in which I have taught you to multiply one root by the other, for two numbers that have a known and visible root, since when multiplying denominated numbers, or roots [= the radicands], one by the other, the root of the product should be extracted, so also one should multiply the root of any number by another root and then extract the root of the product, be it visible or concealed.
|
ועל זה הדמיון אשר למדתיך להכות שרש אחד באחר באלו שני מספרי' אשר להם שרש כי בהכות המספרי' הנקובים או נאמ' שרשים האחד באחר צריך לקחת שרש העולה מההכאה ההיא |
|
שרש ד' פעם שרש ט' עושה שרש מל"ו |
Multiplication of a Root of a Number by a Number |
|
| If you want to multiply a root of a number by a number | ואם רצית לכפול שרש מספר במספר |
|
נניח שרצית לכפול |
| Convert the number into a root: | אז תשיב המספר אל שרש |
|
דהיינו ג' שהוא שרש תשעה |
|
ונאמר אם כן שאנחנו צריכי' להכות שרש ששה בשרש תשעה |
|
אשר למודו הוא להכות ו' על ט' שעושה נ"ד |
|
ושרש נ"ד הוא כפל שרש ששה בשלשה והוא הוא שרש ששה מוכה בשרש תשעה |
|
והנה שרש נ"ד הוא נעלם וכן נבטא בו בנעלם ובמספר בלתי גלוי |
|
רצוני לומר אמרנו |
|
שרש ו' מוכה בג' דהיינו שרש ו' מוכה בשרש ט' עושה שרש נ"ד |
Multiplication of a Root of a Number by a Number and a Root of a Number |
|
| If you wish to multiply a root of a number by a number and by a root of a number: | [9]ואם רצית לכפול שרש מספר במספר ובשרש מספר |
|
נניח שרצית לכפול שרש ה' בשרש ז' וד' |
| You should convert now the number into a root: | עתה אתה צריך להשיב המספר לשרש |
|
דהיינו ד' שהוא שרש לי"ו |
|
ונאמ' א"כ שצריך שנכה שרש ה' בשרש י"ו ובשרש ז' |
|
כי עתה צריך לנו לעשות ה' מוכה בי"ו עושה פ' |
|
כי שרש פ' עולה הכאת שרש ה' |
|
ואח"כ תכפול שרש ה' |
|
דהיינו שאתה צריך לכפול ה' בז' ועושה ל"ה |
|
ושרש זה הל"ה עושה שרש ה' מוכה בז' |
|
אשר שרש ל"ה תשים אצל השרש אשר שמרת הוא שרש פ' ויגיע לידך כי בהכות שרש ה' בד' ושרש ז' עושה |
|
שרש ה' מוכה בד' ושרש ז' עושה שרש פ' ושרש ל"ה |
Multiplication of a Number minus a Root by a Root |
|
| If you wish to multiply a number minus a root by a root: | ואם רצית לכפול מספר פחות שרש בשרש |
|
נניח שרצית לכפול שרש ג' בששה פחות שרש ח' |
| You should now convert the number into a root: | אתה צריך עתה להשיב המספר לשרש |
|
דהיינו ו' ויהיה לך שרש מל"ו |
|
ונאמ' א"כ שאנחנו צריכי' לכפול שרש ג' בשרש ל"ו פחות שרש ח' |
|
שעתה צריכי' אנו |
|
ושרש ק"ח יהיה הכאת שרש ג' בשרש ל"ו אשר זה השרש מק"ח תשמור [ל]חלק אחד מן ההכאה |
|
ואחר תכה שרש ג' בפחות שרש ח' שעולה שרש כ"ד שצריך להוציא משרש ק"ח |
|
וישאר שרש ק"ח פחות שרש כ"ד א"כ בהכות |
Multiplication of a Number and a Root by a Number and a Root - when the numbers are identical and the roots are identical |
|
| If you want to multiply a number and a root by a number and a root: | עוד אם רצית לכפול מספר ושרש במספר ושרש |
|
נניח שרצית לכפול ג' ושרש ה' בג' ושרש ה' |
|
צריך אתה להכות ראשונה המספרי' זה על זה והם ג' על ג' ועולה ט' |
|
אחר כך תכה השרשי' זה על זה שהם שרש ה' בשרש ה' ועולה שרש כ"ה אשר זה השרש הוא ה' |
|
ותקבץ אלו הה' עם ט' ויהיו י"ד מספרי' |
| Then, convert the numbers into a root: | אח"כ תשיב המספרי' לשרשי' |
|
דהיינו כל אחד מהם שהוא ג' ויהיה לך בעד כל אחד מהם שרש ט' |
|
ואלו השרשים תכה בשתי וערב על שרש ה' רצוני שצריך אתה להכות שרש ה' בשרש ט' ואח"כ ג"כ שרש ה' בשרש ט' ויעלה לך בעד כל אחת מההכאות שרש מ"ה ושני השרשי' האלו הם שוים זה לזה |
|
ומקובצי' יחד יעשו שרש מארבעה דמיונם דהיינו שרש מארבעה פעמים |
|
| |
| Know that for every two roots that are summed together, if they are equal, they yield four times their root.
|
ודע כי כל שני שרשי' מקובצי' יחד בהיותם שוים יעשו שרש לארבעה דמיונם |
| From this you seen the proof of the teaching of the addition of unequal roots. | ומזה תראה המופת בלמוד חבור השרשי' הבלתי שוים |
|
ועתה תקבץ זה השרש מק"פ עם שני החלקי' האחרים אשר קבצנו יחד והיו י"ד ויהיה לך י"ד ושרש ק"פ וכן עולה ג' ושרש ה' מוכה בג' ושרש ה' |
| Know that every root of a number multiplied by another identical to it, is the same as saying "its product by itself"; it yields that same number itself, by which the root is denominated [= the radicand].
|
ודע כי כל שרש מספר מוכה באחר שוה לו הוא שוה לאמרך הכאה זו בעצמה ועושה המספר ההוא עצמו אשר ממנו נקרא שרש |
| Therefore, when you multiply 3 plus a root of 5 by 3 plus a root of 5, or any other given identical roots, you only need to add the number by which the root is denominated [= the radicand] to the product of the numbers. | ולזה תכה ג' ושרש ה' בג' ושרש ה' או שרשי' אחרים שיותנו שוים אין לך רק לקבץ על הכאת המספרים המספר אשר שרשו נקוב |
| If the numbers are the same, sum them together, then convert their sum into a root, and multiply this root by one of the roots of the product. | ואם המספרים שוים תקבצם יחד ואחר תשיב [10]הכלל ההוא אל שרש ותכה השרש ההוא |
| Add the root of this product to the sum of the numbers, meaning to the product of the summed numbers. | ושרש זאת ההכאה תקבץ עם המספרי' שקובצו יחד רצוני על הכאת המספרי' שקובצו לשרש |
|
| |
|
והנה המשל נניח שרצונך עוד להכות ג' ושרש ה' בג' ושרש ה' |
|
אתה צריך להכות המספרי' יחד דהיינו ג' בג' ועושה ט' |
|
אח"כ תקבץ עמהם המספר הנקוב אשר לו השרש והוא ה' ויהיה לך י"ד ותשמרם |
|
אח"כ תקבץ המספרי' יחד רצוני ג' וג' ויהיו ו' |
|
ואלו הו' תשיב אל שרש ויהיה לך שרש ל"ו |
|
ושרש ל"ו זה תכה בשרש ה' ויעלה שרש ק"פ |
|
ועתה תקבץ שרש ק"פ על י"ד אשר שמרת ויהיה כללם י"ד ושרש ק"פ וככה תעלה הכאת ג' ושרש ה' בג' ושרש ה' |
| The aforesaid rule is complete. | ונשלם הכלל האמור |
| I inform you that the three aforementioned methods are also written in the next page. | ואודיעך כי שלש השטות הנזכרו' יהיו כתובות ג"כ |
| In order to establish this rule and the one that follows in the next page we brought them here. | ולתקן זה הכלל ואשר ימשך אחריו בדף הנמשך הנחנוהו הנה |
|
והנה לכפול ג' ושרש ה' בג' ושרש ה' יעלה י"ד מספרים ושרש ק"פ |
Multiplication of a Number and a Root by a Number and a Root |
|
| I want to show you also another rule common to all multiplications of numbers and roots either equal or unequal: | עוד רצוני להראותך כלל אחר משותף לכל כפל ממספרי' ושרשי' שוים יהיו או בלתי שוים |
|
ונניח כי בקשת לכפול ג' ושרש ד' בה' ושרש ט' |
| Although you will find this rule in this book below also, since the numbers of the roots are visible, discrete in a foreign language, we present it here. | גם כי תמצא זה הכלל בזה הספר בהמשך אבל מפני כי מספרי השרשים הם גלויים דיסקריטי בלעז נניחהו הנה |
| First, we multiply the numbers by each other. | וראשונה נכפול המספרי' זה נגד זה |
|
רצוני ג' בה' ועולה ט"ו ושמרהו |
| Then, multiply the numbers crosswise by the roots, while converting the numbers into roots: | אח"כ תכפול המספרי' בשרשי' בשתי וערב בהשיב תמיד המספרי' לשרשי' |
|
וא"כ נכה ג' בשרש ט' ויעלה שרש פ"א מפני כי בהשיב ג' אל שרש שיהיה שרש ט' והכות שרש ט' בשרש ט' יעשה ט' שבאמת הוא שרש פ"א |
|
וכן הוא קונבירסו המספר האחר היינו ה' נכהו נגד שרש ד' ועושה שרש ק' |
|
ואלו שתי ההכאות תקבץ עם המספרי' אשר שמרת והיו ט"ו ויהיו לך ט"ו ושרש פ"א ושרש ק' |
| Now, you should multiply the roots by each other. | ועתה צריך אתה לכפול השרשי' האחד על האחר |
|
רצוני שרש ד' בשרש ט' ויהיה לך שרש ל"ו |
|
ותקבצהו אל הסך הראשון ויצא לך בסך כל ההכפלה דהיינו ט"ו ושרש פ"א ושרש ק' ושרש ל"ו שהם במספר גלוי מ' |
| From this rule you can understand all the others whether they involve a visible number or a hidden number. | ומזה הכלל תוכל להבין כל האחרים בין שיהיה להם מספר גלוי או מספר נעלם |
| For the chapter written below was written first at the end of the next page in the context of - "If you wish to multiply a number minus a root by a number minus a root". | ואודיעך כי זה הפרק הכתו' תחת זה הותחל ליכתב בסוף העלה הנמשך האומר ואם רצית להכות מספר פחות שרש במ[ס]פר פחות שרש |
| But, since it was not fully written below as it should have been, and that was a mistake, we present it and explain it properly here. | ובעבור שלא נשלם ליכתב בהמשך כאשר היה ראוי להמשיך וזה היה משגה לכן נניחהו הנה מבואר כראוי |
Multiplication of a Number minus a Root by a Number minus a Root |
|
| I say: if you wish to multiply a number minus a root by a number minus a root: | ואומר אם רצית להכות מספר פחות שרש במספר פחות שרש |
|
נעשה הדמיון שרצית לכפול ג' פחות שרש ה' בד' פחות שרש ז' |
| First, you should multiply the numbers by each other: | אתה צריך ראשונה לכפול המספרי' זה על זה |
|
והם ג' בו' ועולה י"ב ושמרם |
| Then, multiply the numbers by the subtractive roots crosswise; the result is a subtractive root: | אח"כ תכפול המספרי' בשתי וערב בשביל השרשי' שהם פוחתי' [11]ומה שיעלה יהיה שרש פחות |
|
לכן תכה ג' בפחות שרש ז' ועולה שרש ס"ג פוחת |
|
ואחר תכפול ד' בפחות שרש ה' ועולה שורש פ' פוחת |
|
אח"כ תקבץ שני אלו השרשי' יחד שהם פוחתי' ויהיה לך שרש ס"ג פוחת ושרש פ' פוחת |
|
ואלו שני שרשי' הפוחתים צריך להוציאם מן סכום ההכפלה שעושה יותר |
| Now, know that you should multiply the two subtractive roots by each other; the result is an additive root.
|
ועתה דע כי צריך אתה לכפול שני השרשי' הפוחתים זה על זה ויעשה שרש יותר |
| Add this product to the number resulting from the multiplication of the numbers by each other. | וזאת ההכפלה תוסיף על המספר היוצא מהכאת המספרי' זה על זה |
|
א"כ תכה ה' בשרש ז' פוחת ועושה שרש ל"ה יותר |
|
וזה השרש תוסיף על הכפלת המספרי' דהיינו על י"ב ויהיה לך י"ב ושרש ל"ה |
|
עתה תוציא שני השרשי' שאמרנו קודם שהם פוחתי' והם שרש ס"ג ושרש פ' מסכום ההכאה שהיה יותר ויהיה לך י"ב ושרש ל"ה פוחת שרש ס"ג ופוחת שרש פ' וככה עולה הכאת ג' פוחת שרש ה' בד' פוחת שרש ז' |
| Know that the result is the same whether starting by multiplying the roots, then the numbers, or starting with the numbers, then the roots. | ודע כי שוה יצא הדבר להתחיל בהכאת השרשים ולהשלים במספרי' כמו בהתחיל במספרי' ולהשלים בשרשי' |
| Remember always to sum the additive products together, before starting to subtract a number or a root from any product, in order to have a true understanding of the stated multiplication, or others that will be carried out. | וזכור לעולם להוסיף עליו ההכאות שעושות יותר יחד קודם שתתחיל להוציא המספר או איזה |
|
ג' פחות שרש ה' בד' פחות שרש ז' עושה י"ב ושרש ל"ה פחות שרש ס"ג ופחות שרש פ' |
| I also want to show you another rule common to all [types of] multiplications of numbers and roots either equal or unequal: | עוד רצוני להראותך כלל אחר משותף לכל כפל בין יהיה שוה ממספרי' ומשרשי' או לא יהיה שוה |
|
נניח שרצונך לכפול ג' ושרש ה' בד' ושרש ז' |
| First, you should multiply the numbers by each other: | אתה צריך ראשונה לכפול המספרים יחד |
|
דהיינו ג' בד' ועולה י"ב |
|
אח"כ תכפול המספרי' בשרשים בשתי וערב בהשיב תמיד המספרי' לשרשים |
|
לכן תכפול ג' בשרש ז' שעולה שרש ס"ג |
|
ואח"כ תכפול ד' בשרש ה' עולה שרש פ' |
|
ושני אלו השרשי' תקבץ אל המספרי' אשר שמרת והיו י"ב ויהיה לך י"ב ושרש ס"ג ושרש פ' |
| Now, multiply the two roots by each other: | ועתה תכפול השרשים זה על זה |
|
רצוני שרש ה' בשרש ז' ועולה שרש ל"ה |
|
וזה תחבר אל הסכום הראשון ויהיה לך סך |
| Know that the result is the same whether starting by multiplying the roots, or starting with the numbers. | ודע כי ככה ישוה |
| Know also that multiplying one of the numbers by the number and the root of the other term, then multiplying the root given in the term of that number by the number and the root of the other term, and summing all the products together - is the same as the multiplication in the ways mentioned above. | עוד דע כי ככה שוה לכפול אחד מהמספרי' במספר אשר בצד האחר ובשרש ואחר תכפול השרש המונח מהמספר במספר ובשרש מהצד האחר ואח"כ תקבץ כל דבר יחד כמו בכל אחד מהאופני' הנזכרי' בזה האופן בכפול הכפל הכתו' למעלה |
|
תכפול עתה ג' בד' ושרש ז' ועולה י"ב ושרש ס"ג |
|
ואחר תכפול השרש המונח מהמספר והוא שרש ה' בד' ושרש ז' עולה שרש פ' ושרש ל"ה ותהיה נשלמת ההכפלה הנזכרת |
|
ג' ושרש ה' בד' ושרש ז' עולה י"ב ושרש ס"ג ושרש פ' ושרש ל"ה |
| Also, if you wish to multiply a number minus a root by a number minus a root: | [12]והנה עוד אם בקשת לכפול מספר פחות שרש במספר פחות שרש |
|
נניח שרצית לכפול ג' פחות שרש ה' בד' פחות שרש ז' |
| You should multiply the numbers by each other. | אתה צריך לכפול המספרי' זה בזה |
|
דהיינו ג' בד' ועולה י"ב ושמרהו |
| Know that this rule is given in the previous page, since it does not continue here. | דע כי זה הכלל הושם בעלה |
| Also, I want to show you that in multiplication of numbers a subtractive by subtractive generates an additive.
|
עוד רצוני להראותך במספר כי פחות בפחות יעשה יותר בהכפלה |
| Since every time you wil multiply a subtractive by a subtractive you will clearly see that it yields an additive. | בעבור כי בכל פעם שיזדמן לך לכפול פחות בפחות תראה מבואר שיעשה יותר |
|
והנה המשל ח' מוכה בח' עושה ס"ד |
|
וח' הוא ב' פחות מי' |
|
ולהכותו בח' אחר שהוא ג"כ פחות מי' ראוי שיעלה כדומה לו ס"ד |
|
ולזה נאמ' שי' פחות ב' מוכה בי' פחות ב' ראוי שיעשה ס"ד |
|
וראית זה כי בהכותנו י' על י' עושה ק' |
|
וי' מוכה בב' פחות עושה כ' פחות דהיינו י' בב' שהוא מהצד האחר |
|
עתה תכה בשתי וערב י' שהוא בצד האחר בב' האחר שהוא ג"כ פוחת עושה ג"כ כ' פוחת |
|
ועתה תקבץ אלו שתי ההכאות הפוחתות יחד ויעלו מ' פוחתים |
|
והוצא אלו המ' הפוחתי' מכפל י' בי' שהוא ק' וישאר ס' |
|
עתה חסר להשלים ההכפלה להכות ב' פוחתי' בב' פוחתי' אשר אומר שעושה ד' יותר |
|
אשר בהוסיפו על ס' עושה היטב ס"ד וככה עולה לכפול י' פחות ב' בי' פחות ב' דהיינו ח' בח' |
|
ולכן אם פחות מוכה בפחות לא היה עושה כלום היה ראוי להוציא ד' או לקבצם מס' א"כ ימשך כי י' פחות ב' מוכה בי' פחות ב' דהיינו ח' בח' יעשה ס' וזה יהיה כזב |
|
| |
|
ואם פחות מוכה בפחות רצוני אלו הב' פוחתים מוכים בב' פוחתי' יעשה ד' פוחתי' זה הד' יצטרך לגרעו מס' וישאר נ"ו |
|
| |
| Hence, a subtractive multiplied by a subtractive is necessarily additive.
|
א"כ פחות מוכה בפחות מחויב הוא שיעשה יותר |
|
י' פחות ב' בי' פחות ב' עולה ס"ד |
Multiplication of a Number minus a Root by a Number minus a Root - when the numbers are identical and the roots are identical |
|
| If you wish to multiply a number minus a root by a number minus a root, when the numbers are identical and the roots are identical: | ואם רצית לכפול מספר פחות שרש במספר פחות שרש בהיות המספרי' שוים והשרשי' זה לזה |
|
ונניח שרצית לכפול ג' פחות שרש ה' בג' פחות שרש ה' |
| You should multiply the numbers by each other: | הנך צריך להכות המספרי' זה על זה |
|
דהיינו ג' בג' ועולה ט' |
|
ועל מספר זה מט' תוסיף המספר מאחד השרשים שהוא ה' ויהיו י"ד ותשמרם |
|
אח"כ תקבץ המספרי' יחד דהיינו ג' וג' ויהיו ו' |
|
וזה הו' תביא אל שרש ויהיה לך שרש ל"ו |
|
ותכפול שרש ל"ו באחד השרשים שהוא שרש ה' שהוא פחות ועולה שרש ק"פ פוחת |
|
ושרש ק"פ זה תוציא ממספר י"ד אשר שמרת וישאר י"ד פחות שרש ק"פ וככה עולה כפל ג' פחות שרש ה' בג' פחות שרש ה' |
| Also, if you want to multiply these or similar ones by another method of multiplying a number and a root by a number and a root - as I have shown you previously, you should do as follows: | עוד אם רצית לכפול כפל זה או דומה לו באופן אחר אשר הראיתיך לפנים לכפול מספר ושרש במספר ושרש ככה ראוי לך לעשות |
|
כפול ראשונה המספרי' זה על זה |
|
דהיינו ג' על ג' ועולה ט' |
|
אח"כ תכה [13]עוד העושה יותר דהיינו שרש ה' הפוחת בשרש ה' הפוחת שהוא |
|
וקבץ זה הה' עם כפל המספרי' שהיה ט' ויעלה י"ד ותשמרם |
|
ועתה תכה ג' בפחות שרש ה' בשתי וערב ויהיה לך שרש מ"ה לכל אחת מההכאות שהוא פוחת |
|
ולכן יהיה לך ב' פעמי' שרש מ"ה פוחת ולזה תכה ב' בשרש מ"ה ועולה שרש ק"פ פוחת |
|
ושרש ק"פ זה תוציא מי"ד אשר שמרת וישאר י"ד פחות שרש ק"פ וככה עולה הכאת ג' פחות שרש ה' בג' פחות שרש ה' |
| You can carry out this type of multiplication also by the aforementioned methods. | אשר הכאה זו תוכל ג"כ לעשותה באופני' האמורי' למעלה |
|
ג' פחות שרש ה' בג' פחות שרש ה' עושה י"ד פחות שרש ק"פ |
Multiplication of a Number and a Root by a Number minus a Root - when the numbers are identical and the roots are identical |
|
| If you wish to multiply a number plus a root by a number minus a root: | ואם רצית לכפול מספר ושרש במספר פחות שרש |
|
נניח שרצית לכפול ה' ושרש ג' בה' פחות שרש ג' |
| You should multiply the numbers by each other: | אתה צריך להכות המספרי' זה על זה |
|
דהיינו ה' על ה' ועולה כ"ה ותשמרם |
| Now, multiply the additive root by the number on the other side: | עתה תכה השרש שהוא יותר במספר שהוא בצד האחר |
|
דהיינו שרש ג' בה' ועולה שרש ע"ה |
| Then, multiply the subtractive root by the number on the other side: | [אח"כ תכה [השרש הפוחת] במספר [אשר בצד האחר] |
|
דהיינו [שרש ג' בה' ועולה] שרש מע"ה][14] וזה השרש בא להיות פוחת |
| Since a subtractive multiplied by an additive yields a subtractive.
|
בעבור כי פחות במוסיף עושה פחות |
| The subtractive should be subtracted from the additive root, but since they are equal - nothing remains. | וזה הנפחת צריך להוציא מהשרש המוסיף ומפני היותם שוים ישאר לא כלום |
|
ולכן לא ישאר עד עתה מהכפל רק אשר היה מכפל המספרי' זה על זה דהיינו כ"ה |
I remind you that whenever you multiply a number and a root that are the same as the number minus the root on the other term [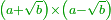 ], you do not have to multiply the numbers by the roots, because the subtractive cancels the additive, as they are equal. ], you do not have to multiply the numbers by the roots, because the subtractive cancels the additive, as they are equal.
|
ולכן אזכירך שבכל פעם שיבואך הכאת מספר ושרש שוים למספר פוחת שרש בצד האחר אינך צריך להכות המספרי' בשרשי' בעבור כי הפוחת ממעיט המוסיף מפני השואתם |
| Therefore, the only thing left for you to do in [this type of] multiplication is to multiply the additive root by the subtractive root.
|
ולכן אין צריך לך לעשות מהכפל עתה רק להכות השרש שהוא מוסיף בשרש שהוא פוחת |
|
דהיינו שרש ג' המוסיף בשרש ג' הפוחת העושה שרש ט' פוחת שהוא ג' |
|
וזה הג' תוציא מכ"ה וישאר כ"ב וככה עולה הכאת ה' ושרש ג' בה' פחות שרש ג' |
|
ה' ושרש ג' בה' פחות שרש ג' עולה כ"ב |
Multiplication of a Number and a Root by a Number minus a Root |
|
| I also want to show you another rule common to all [types of] multiplications of numbers and roots either equal or unequal, when one term is additive and the other term is subtractive: | עוד רצוני להראותך כלל אחר משותף לכל כפל שוה יהיה ממספר ושרש או לא יהיה שוה בהיות יותר מצד אחד ופחות מהאחר |
|
נניח שרצית לכפול ג' ושרש ד' בה' פחות שרש ט' |
| You should multiply the numbers by each other. | הנך צריך עתה להכות המספרי' זה על זה |
|
דהיינו ג' בה' ועולה ט"ו ושמרם בצד אחד |
| Then, multiply by the number of the other term. | אח"כ תכה במספר מהצד האחר |
|
רצוני שרש ד' בה' ועולה שרש ק' |
|
הוסיפהו על כפל המספרי' זה על זה שהוא ט"ו ויהיה לך ט"ו ושרש ק' |
| Now, multiply the subtractive root by the number of the other term: | עתה תכה השרש הפוחת במספר הצד האחר |
|
דהיינו שרש ט' הפוחת בג' ועולה שרש פ"א הפוחת |
| Keep the subtractive root to be subtracted from the sum we produced above. | ותשמור להוציא זה השרש הפוחת מהסכום שעשינו למעלה |
| Then, multiply the subtractive root by the additive root of the other term:
|
אח"כ תכה השרש הפוחת מצד אחד בשרש המוסיף מהצד האחר |
|
שהוא שרש ט' הפוחת בשרש ד' המוסיף ויהיה לך שרש ל"ו פוחת |
| Subtract these two subtractive roots from the sum above. | ואלו שני השרשי' הפוחתי' תוציא מהסכום אשר למעלה |
|
וישאר ט"ו ושרש ק' פוחת שרש פ"א ופחות שרש ל"ו |
| The reason that we operate in this rule according to the way of the numbers whose roots are known is that we do it in order that the rule will be common to the known roots as well as the unknown roots. | [והסבה][15] בהיות כי אנחנו פעלנו בזה הכלל על דרך השרשי' [16]והמספרי' הנקובים להם שרשים' ידועים הנה עשינו זה כדי שהכלל יהיה משותף קומונו בלעז לשרשי' ידועי' ולבלתי ידועי' |
|
בהיות כי בהכות ג' ושרש ד' שהוא ב' בה' פחות שרש ט' שהוא ג' ישאר ב' [ונכה ב' בה'][17] ועולה עשרה |
|
וג"כ ככה עולה ההכאה של מעלה שהיא |
|
ט"ו מחובר עם שרש ק' שהוא עשרה ועושה כ"ה |
|
ובהוציאנו מזה שני השרשי' הגורעי' שהם שרש פ"א שהוא ט' ושרש ל"ו שהוא ו' שהם עולים ט"ו מאלו הכ"ה וישארו עשרה הכפל ההוא וככה עולה ג' ושרש ד' מוכה בה' פחות שרש ט' |
|
| |
|
ג' ושרש ד' בה' פחות שרש ט' עולה עשרה |
Multiplication of a Root by a Root minus a Number |
|
| Know also, if you wish to multiply a root by a root minus a number: | ודע עוד אם רצית לכפול שרש בשרש פחות מספר |
|
נניח שרצית לכפול שרש ח' בשרש ח' פחות ב' |
| You should multiply the roots by each other: | הנך צריך להכות השרשי' זה על זה |
|
רצוני שתכה שרש ח' בשרש ח' ועושה ח' |
| Since the roots are equal, it is the same as if you multiply one of them by itself, meaning that it yields the number by which the root is denominated [= the radicand].
|
מפני שהשרשי' שוים ושוה הדבר כאלו כפלת אחד מהם בעצמו רצוני שראוי שיעשה המספר הנקוב שהוא לו שרש |
| If the roots are not identical, we say that this product is the root of the product of the numbers, by which the roots are denominated [= the radicands], one by the other.
|
ואם השרשי' לא יהיו שוים אנחנו נאמ' שהכפל יהיה שרש הכפל אשר יעשה מהכאת המספרי' הנקובי' להיות להם שרשי' זה בזה |
|
ולכן נאמ' אנחנו שיעשה שרש מס"ד שהוא מהכאת ח' בח' ושמור זה הח' שהוא שרש מס"ד |
| Then, multiply the subtractive number by the root of the first term: | ואח"כ תכפול המספר הפוחת משרש החלק האחד |
|
דהיינו ב' בשרש החלק האחר שהוא בשרש ח' ועושה שרש מל"ב הפוחת |
|
ולכן תוציא שרש מל"ב הפוחת חוץ |
| If the roots are not identical, or if the product of the numbers, whose roots are multiplied by each other, has no root, you should convert them into a root minus a root. | ואם השרשים לא היו שוים או כי בהכאת המספרי' אשר הם להם שרשי' [זה על זה][18] לא יהיה לה שרש אתה צריך להשיב שרש פחות שרש |
|
כאלו תאמר שרש ס"ד פחות שרש ל"ב וככה עולה הכאת שרש ח' בשרש ח' פחות ב' |
|
שרש ח' מוכה בשרש ח' פחות ב' עולה ח' פחות שרש ל"ב |
Multiplication of a Root minus a Number by a Root minus a Number |
|
| If you wish to multiply a root minus a number by a root minus a number: | ואם רצית לכפול שרש פחות מספר בשרש פחות מספר |
|
ונניח שרצית לכפול שרש ח' פחות ב' בשרש עשרה פחות ג' |
| You should multiply the roots by each other: | אתה צריך לכפול השרשי' זה על זה |
|
רצוני שרש ח' בשרש עשרה ועולה שרש פ' ושמרהו |
| Then, multiply the subtractive numbers of each term: | אח"כ תכפול המספרי' הפוחתי' מכל אחד מהחלקי' |
|
שהם ב' פוחתי' בג' פוחתי' ועושים ו' מוספי' |
|
תוסיף זה הו' על שרש פ' אשר שמרת ויהיה לך שרש פ' וו' יותר |
| Then, multiply the subtractive numbers by the counter roots crosswise: | אח"כ תכפול המספרים שהם גורעי' בשרשי' שהם מנגדי' שתי וערב |
|
דהיינו ב' הפוחתי' מהצד האחד בשרש אשר בצד האחר ועולה שרש מ' הפוחת |
|
אח"כ תכה המספר האחר הפוחת שהוא ג' פחות שרש ח' אשר הוא מהצד האחר ויהיה לך שרש ע"ב הפוחת |
|
עתה תוציא שני אלו השרשי' הפוחתי' מהסכום של מעלה שהוא שרש מ' ושרש ע"ב וישאר ו' ושרש פ' פחות שרש מ' ופחות שרש ע"ב וככה עולה כפל שרש ח' פחות ב' בשרש עשרה פחות ג' |
| Know that starting by multiplying the subtractive numbers first is the same as starting by multiplying the roots first. | ודע כי הדבר שוה בהתחיל לכפול ראשונה מן המספרי' הגורעי' כמו בהתחיל [19]לכפול ראשונה מהשרשי' |
| Always remember to multiply first the multiplicands whose products are additive in order to sum up all that should be added in the product, then to subtract all that should be subtracted, as you did in the multiplication above. | ותזכור לעולם לכפול ראשונה חלקי הכפל אשר מוכפלים יעשו יותר בסבת חבר |
|
שרש ח' פחות ב' בשרש י' פחות ג' עולה ו' ושרש ח' גורע שרש מ' ופחות שרש ע"ב |
Multiplication of a Root minus a Number by a Root minus a Number - when the numbers are identical [and the roots are identical] |
|
| If you wish to multiply a root minus a number by a root minus a number, when the numbers are identical [and the roots are identical]: | עוד אם בקשת לכפול שרש פחות מספר בשרש פחות מספר בהיות המספרי' שוים זה לזה |
|
ונניח שרצית לכפול שרש י"ב פחות ב' בשרש י"ב פחות ב' |
| First you should multiply the roots by each other: | אתה צריך להכות ראשונה השרשי' זה בזה |
|
דהיינו שרש י"ב בשרש י"ב ועולה י"ב |
| Then, multiply the subtractive numbers by each other: | ואח"כ תכפול המספרי' הפוחתי' זה על זה |
|
דהיינו ב' פוחתי' בב' פוחתי' ועושי' ד' יותר |
|
ותקבץ עם י"ב ויהיה לך י"ו ושמרם |
| Then, sum up the numbers together: | ואח"כ קבץ המספרי' יחד |
|
דהיינו ב' פוחתים עם ב' פוחתי' ויהיו לך ד' פוחתי' |
|
וזה הד' תכפול באחד מהשרשי' רצוני בשרש י"ב ויהיה לך שרש מקצ"ב פוחת |
|
וזה השרש תוציא מי"ו וישאר י"ו פחות שרש מקצ"ב וככה עולה כפל שרש י"ב פחות ב' בשרש י"ב פחות ב' |
| We can perform this multiplication by another method: | עוד נוכל לעשות זה הכפל באופן אחר |
|
אחר שכפלת שרש י"ב בשרש י"ב שעושה י"ב |
|
ואח"כ ב' פוחתי' בב' פוחתי' שעושה ד' יותר |
|
ומקובצי' יחד עושה י"ו |
| You then multiply each root by the counter subtractive number crosswise: | ואחר תכפול כל שרש עם המספר המקביל לו הפוחת בשתי וערב |
|
רצוני שרש י"ב בב' הפוחת ועושה שרש מ"ח פוחת |
|
ואח"כ השרש האחר מי"ב בב' האחר הפוחת אשר מצד האחר ועולה שרש ממ"ח פוחת |
|
אשר יהיה בין שני כפלי שרשי מ"ח פוחתי' שרש מקצ"ב פוחתי' |
| You have this [type] of multiplication performed by the two methods. | ויהיה לך הכפל הזה עשוי בשני האופני' |
|
והוא י"ו פחות שרש קצ"ב |
|
שרש י"ב פחות ב' בשרש י"ב פחות ב' עולה י"ו פחות שרש קצ"ב |
Multiplication of a Root minus a Number by a Root and a Number |
|
| If you wish to multiply a root minus a number by a root plus a number: | עוד אם רצית לכפול שרש פחות מספר בשרש ומספר |
|
נניח שרצית לכפול שרש ט"ו פחות ג' בשרש י"ב וב' |
| You should multiply the roots by each other: | עתה צריך אתה לכפול השרשי' זה בזה |
|
דהיינו שרש ט"ו בשרש י"ב ועולה שרש ק"פ ושמרהו |
| Now, multiply the additive number on one side by the root on the other side: | עתה תכפול המספר היותר מהצד האחד בשרש אשר בצד האחר |
|
דהיינו ב' בשרש ט"ו ועולה שרש מס' |
|
וקבצהו עם שרש ק"פ אשר שמרת ויהיה לך שרש ק"פ ושרש ס' ושמרם |
| Then, multiply the subtractive number on one side by the root on the other side: | אח"כ תכפול המספ' הפוחת מצד אחד בשרש אשר מצד אחר |
|
דהיינו ג' פוחתי' בשרש י"ב ועולה שרש מק"ח הפוחת |
| Multiply also the subtractive number and the additive [number] by each other: | ועוד תכפול המספרי' הפוחתי' והיותר מהשרשי' האחד באחר |
|
דהיינו ג' הפוחתי' בב' המוסיפי' ועולי' ו' פוחתי' |
|
עתה הוצא אלו שתי ההכפלות הפוחתים שהם שרש מק"ח הפוחת וו' הפוחתי' מהסכום הנז' שהוא שרש מק"פ ושרש מס' וישאר שרש מק"פ ושרש מס' פחות שרש מק"ח פחות ו' |
| Always remember to multiply first the multiplicands whose products are additive, then multiply those [whose products are] subtractive, in order to subtract them from the additive. | וזכור תמיד לכפול [20]ראשונה כל החלקי' מההכפלו' אשר בהכפלם יעשו יותר ואח"כ תכפול העושים פחות להוציאם מהרב |
|
והנה בכפול שרש ט"ו פחות |
Multiplication of a Root and a Number by a Root minus a Number - when the numbers are identical and the roots are identical |
|
| If you wish to multiply a root plus a number by a root minus a number, when the numbers are identical and the roots are identical. | עוד אם רצית לכפול שרש ומספר בשרש פחות מספר בהיות המספרי' שוים זה לזה והשרשי' זה לזה |
|
ונניח |
| First you should multiply the roots by each other: | הנך צריך ראשונה לכפול השרשי' זה בזה |
|
דהיינו שרש ח' בשרש ח' ועולה ח' ושמרם |
| Then, we continue to multiply the root on one side by the subtractive number on the other side. | אח"כ נמשיך לכפול השרשי' אשר בצד אחד במספר אשר פוחת בצד האחר |
| Afterwards the additive number on one side by the root on the other side. | ואח"כ המספר אשר בצד אחד שהוא יותר בשרש אשר בצד האחר |
| Since these two products are equal to one another, but one is additive and the other is subtractive, when they are summed together it yields nothing. | ובעבור שתי אלה ההכפלות שהם שוות זו לזו עושה יותר והאחרת עושה פחות בחברם יחד עושה לא כלום |
| Therefore nothing should be done in this multiplication and its similar, except for multiplying the subtractive number by the additive number. | ולכן לא יצטרך בכפל הזה |
|
דהיינו בזה ב' פחות ב' בב' יותר שהוא עושה ד' פוחתי' |
|
והוצא מכפל השרשי' זה בזה שהוא ח' וישאר ד' וככה עולה כפל שרש ח' וב' בשרש ח' פחות ב' רצוני שעולה ד' |
Multiplication of a Root by a Root and a Root |
|
| If you wish to multiply a root by a root plus a root. | ואם רצית לכפול שרש בשרש ושרש |
|
ונניח שרצית לכפול שרש ה' בשרש ז' ושרש עשרה |
| You should multiply the single root by each of the two other roots, or likewise if there were more, in the way we multiply a root by a root. | הנך צריך לכפול השרש היחיד באחד אחד מאותם השנים באופן אשר נכפול שרש בשרש וכן אם היו יותר בזה האופן |
|
תכפול ראשונה שרש ה' בשרש ז' שעולה שרש ל"ה ושמרהו |
|
אח"כ תכפול עוד שרש ה' בשרש עשרה ועולה שרש נ' |
|
וקבצם יחד ויהיה לך שרש ל"ה ושרש נ' וככה עולה הכאת שרש ה' בשרש ז' ושרש עשרה |
Multiplication of a Root by a Root minus a Root |
|
| If you wish to multiply a root by a root minus a root. | ואם רצית להכות שרש בשרש פחות שרש |
|
ונניח שבקשת לכפול שרש ה' בשרש י"ב פחות שרש ח' |
|
הנך צריך לכפול ראשונה שרש ה' בשרש י"ב שעולה שרש ס' ושמרם |
|
אח"כ תכפול שרש ה' בשרש הפוחת שהוא שרש ח' ועולה שרש מ' פוחת |
|
ועתה הוצא הכפל הזה הגורע מהכפל אשר שמרת וישאר שרש ס' פחות שרש מ' וככה עולה הכאת שרש ה' בשרש י"ב פחות שרש ח' |
Multiplication of Two Roots by Two other Roots |
|
| Likewise, if you wish to multiply two roots by two other roots. | עוד אם רצית לכפול ב' שרשים בב' שרשים אחרים |
|
ונניח שבקשת לכפול שרש ה' ושרש ז' בשרש עשרה ושרש ט"ו |
| Multiply these roots in the aforementioned way of multiplying numbers and roots: | תכפול אלו השרשי' באופן האמור בהכאת מספרי' ושרשי' |
| First, start to multiply the first counter roots: | וראשונה תתחיל לכפול מהשרשי' הראשוני' המתנגדי' |
|
דהיינו שרש ה' בשרש עשרה ועולה שרש נ' ושמרם |
| Multiply the first counter roots by the other roots crosswise: | אח"כ תכפול בשתי וערב השרשי' הראשוני' המתנגדי' בשרשי' השניים |
|
דהיינו שרש ה' בשרש ט"ו ועולה שרש ע"ה |
|
אח"כ תכה שרש עשרה בשרש ז' ועולה שרש ע' |
|
וקבץ שתי אלו ההכאות עם הראשונה ויהיו לך שרש נ' ושרש ע' |
| Then, multiply the two last counter roots by each other: | אח"כ תכפול שני השרשי' האחרוני' המתנגדי' זה על זה |
|
דהיינו [21]שרש ז' בשרש ט"ו ועולה שרש ק"ה |
|
וקבצם עם הכאות שלשת השרשי' האחרים והיה לך שרש נ' ושרש ע"ה ושרש ע' ושרש ק"ה כלם יחד וככה עושה להכות שרש ה' ושרש ז' בשרש עשרה ושרש ט"ו |
| If you wish to start multiplying from the later roots apply the way of multiplying numbers crosswise in boxes casillas in foreign language. | ואם בקשת להתחיל לכפול מן השרשי' האחרוני' תהיה רודף אופן כפל [המספרים בדרך][22] הבתי' קאסילי בלעז בשתי וערב |
| You can write the multiplication of the roots in the way the numbers are written. | וכן תוכל לכתוב כפל השרשי' באופן שבאי' לכתוב המספרים |
|
שרש ה' ושרש ז' בשרש י' ושרש ט"ו עולה שרש נ' ושרש ע"ה ושרש ע' ושרש ק"ה |
Multiplication of Two Roots by Two other Roots - when the first roots of both parts are identical and the second roots of both parts are identical |
|
| If you wish to multiply a root plus a root by a root plus a root, when the later and the former numbers are identical. | אם בקשת לכפול שרש ושרש בשרש ושרש בהיות שוים המספרי' השניים והראשוני' |
|
ונניח שרצית לכפול שרש ה' ושרש ז' בשרש ה' ושרש ז' |
|
הנך צריך ראשנה אם באת לפעול בדרך הקאסילי לכפול שרש ז' בשרש ז' שעולה ז' ושומהו |
|
אח"כ תכפול בשתי וערב שרש ז' בשרש ה' עולה שרש ל"ה |
|
ואח"כ תכפול שרש ז' האחר בשתי וערב בשרש ה' האחר ויהיה עוד לך שרש ל"ה |
|
ואלו שני השרשי' תקבץ עם הז' ששמרת ויהיה לך שני פעמי' שרש ל"ה שהוא שרש ק"מ וז' יותר היו בידך אשר אשר תקבצם עמהם |
|
ואח"כ תכפול שרש ה' בשרש ה' ועולה ה' |
|
וזה הה' תקבץ עם הסך האמור ויהיה לך י"ב ושרש ק"מ |
|
ואפע"פ שכפלנו בדרך הקסילי לא עלה לנו באופן ההוא מפני כי הכפל הראשון קובץ עם השני להשיב ראשונה המספרי' ואח"כ השרשי' |
|
שרש ה' ושרש ז' בשרש ה' ושרש ז' עולה שרש ק"מ וי"ב מספרי' |
Multiplication of a Root and a Root by a Root minus a Root |
|
| If you wish to multiply a root plus a root by a root minus a root: | ואם רצית לכפול שרש ושרש בשרש פחות שרש |
|
ונניח שרצית לכפול שרש ה' ושרש ז' בשרש עשרה פחות שרש ו' |
|
תכפול ראשונה שרש ה' בשרש עשרה ועולה שרש נ' |
|
אח"כ תכה שרש שרש ז' שהוא יותר בשתי וערב בשרש עשרה ועולה שרש ע' |
|
וקבץ יחד ויהיה לך שרש נ' ושרש ע' |
|
אח"כ תכה בשתי וערב שרש ו' הפוחת ועושה שרש ל' הפוחת |
|
ואח"כ תכה שרש ו' הפוחת בשרש ז' המוסיף ועולה שרש מ"ב פוחת |
|
ואלו שתי ההכאות הפוחתי' הוצא מהשתי הכאות ראשונות שעשית וישאר שרש נ' ושרש ע' פחות שרש ל' ופחות שרש מ"ב וככה עולה להכות שרש ה' מקובץ עם שרש ז' בשרש עשרה פחות שרש ו' |
Multiplication of a Root and a Root by a Root minus a Root - when the first roots of both parts are identical and the second roots of both parts are identical |
|
| If you wish to multiply a root plus a root by a root minus a root, when the first of the former is the same as the first of the [later] and the second of the former is the same as the second of the later. | עוד אם רצית לכפול שרש ושרש בשרש פחות שרש בהיות שוים הראשון מהחלק הראשון לראשון מהחלק הראשון והשני מהחלק ראשון לשני מהחלק השני |
|
ונניח שרצית לכפול שרש עשרה ושרש ז' בשרש עשרה פחות שרש ז' |
| First, you should multiply the roots by each other: | הנך צריך ראשונה לכפול השרשי' זה בזה |
|
דהיינו שרש עשרה בשרש עשרה ועולה עשרה ושמרם |
| Then, you only need to multiply the other roots by each other: | אח"כ אין לך לכפול רק השרשי' האחרוני' זה בזה |
|
דהיינו שרש ז' בפחות שרש ז' ועולה ז' הפוחת |
|
והוצא זה הז' הפוחת מעשרה אשר שמרת [23]וישאר שלשה וככה עולה לכפול שרש עשרה ושרש ז' בשרש עשרה פחות שרש ז' |
| Remember that in this calculation and in similar [calculations] there is [no] need to multiply the roots crosswise, since they are the same - one is subtractive and the other is additive. | וזכור כי בזה החשבון ובדומי' אליו צריך לכפול השרשי' בשתי וערב בהיותם שוים בהיות האחד פוחת והאחר מוסיף |
| Because one multiplication cancels the other, as one yields a subtractive and the other an additive. | מפני כי הכפל האחד מכשיל האחר כי האחד עושה פחות והאחר יותר |
|
והנה בכפול שרש עשרה ושרש ז' בשרש עשרה פחות שרש ז' עולה ג' מספרים |
| The same is done with all those that are similar to them. | וכזה יעשה לכל הדומי' אליו |
Multiplication of a Root minus a Root by a Root minus a Root |
|
| If you wish to multiply a root minus a root by a root minus a root. | עוד אם רצית לכפול שרש פחות שרש בשרש פחות שרש |
|
נניח שרצית לכפול שרש י"ב פחות שרש ז' בשרש ט"ו פחות שרש עשרה |
| First, you should multiply the additive roots by each other: | תצטרך ראשונ' לכפול |
|
דהיינו שרש י"ב בשרש ט"ו ועולה שרש ק"פ ושמרהו |
| Then, multiply the additive roots by the subtractive roots crosswise: | אח"כ תכפול השרשי' המוסיפי' בשרשי' הגורעי' בשתי וערב |
|
דהיינו שרש י"ב בפחות שרש עשרה ועולה שרש ק"כַ שהוא פחות |
|
אח"כ תכפול שרש ט"ו בפחות שרש ז' ועולה שרש ק"ה שהוא פוחת |
| Set these two subtractive roots aside. | ושים שני אלו השרשי' הפוחתי' לבד |
| Then, multiply the subtractive roots by each other: | ואח"כ תכפול השרשי' הפוחתי' זה בזה |
|
דהיינו שרש ז' בשרש עשרה ועולה שרש ע' שהוא יותר |
|
|
|
עתה הוצא שני השרשי' הפוחתי' מזה הסך וישאר שרש ק"פ ושרש ע' פחות שרש ק"כ ופחות שרש ק"ה וככה עולה הכפל הנז' |
Multiplication of a Root minus a Root by a Root minus a Root - when the first roots of both parts are identical and the second roots of both parts are identical |
|
| If you wish to multiply a root minus a root by a root minus a root, when the first [of the former] is the same as the first [of the later] and the second [of the former] is the same as the second of the later. | עוד אם רצית לכפול שרש פחות שרש בשרש פחות שרש בהיות שוים השרשי' הראשוני' לראשוני' והשניים לשניים מהחלק האחר |
|
ונניח שרצית לכפול שרש י"ב פחות שרש ז' בשרש י"ב פחות שרש ז' |
| First, you should multiply the [additive] roots by each other: | אתה צריך ראשונה לכפול השרשי' הרבי' זה בזה |
|
דהיינו שרש י"ב בשרש י"ב ועולה י"ב ושמרם |
| Then, multiply the [subtractive] roots by each other: | אח"כ תכפול השרשי' שהם מעטי' זה בזה |
|
דהיינו הפוחת שרש ז' בפוחת שרש ז' ועולה ז' יותר |
|
וקבצם יחד עם אשר שמרת ויהיה לך י"ט ושמרם |
| Then, multiply the additive roots by the [subtractive] roots crosswise: | אח"כ תכה השרשי' שהם יותר בשרשי' שהם מעט בשתי וערב |
|
דהיינו שרש י"ב בשרש ז' גורעי' ושרש ז' הגורע עוד בשרש י"ב ויהיה לך ב' פעמי' שרש פ"ד אשר שני אלו השרשי' הם ב' |
|
הוציאם מהסך ששמרת שהוא י"ט וישאר לך י"ט פחות שרש של"ו וככה עולה לכפול שרש י"ב פחות שרש ז' בשרש י"ב פחות שרש ז' |
Multiplication of a Number by a Root |
|
| Remember that whenever you happen to multiply a number by a root, you should convert the number into a root of the same degree - whether a square root, or a cube root, or any other degree. | וזכור כי בכל פעם שיגיע בידך לכפול מספר באיזה שרש צריך שתשיב המספר למין השרש אשר אתה רוצה לכפול מרובע או מעוקב או בכל אופן שיוכל להגיע |
| If you happen to multiply a root by another root of a different degree [lit. not similar to it by nature], you should convert each of the roots to the root of the parallel degree. | ואם יגיע בידך לכפול איזה שרש בשרש אחר אינו דומה אליו בטבע אתה צריך להשיב כל אחד ממספרי השרשי' ההם אל שרש המספר המתנגד לטבע |
|
והנה המשל מהמספר בשרשי' בכפול מספר בשרש מרובע צריך לכפול המספר בעצמו ואח"כ תכפול העולה ממנו במספר השרש האחר והעולה מזה הנה שרשו הוא יהיה הכאתו כאשר יתבאר לפנים בזה האופן |
|
ונניח שבאת [24]לכפול ג' בשרש מרובע מד' |
|
הנך צריך להשיב ג' לשרש מרובע שהוא שרש מט' |
|
ותכפול ד' בט' שעושה ל"ו |
|
ושרש ל"ו שהוא ו' הוא הכפל האמור |
|
ואם תכפול ג' בשרש מעוקב מח' |
|
אתה צריך להשיב ג' לשרש מעוקב שהוא יהיה שרש מעוקב מכ"ז |
|
ותכפול ח' בכ"ז ועולה רי"ו |
|
ושרש מעוקב מרי"ו שהוא ו' הוא הכפל האמור |
Multiplication of a Square Root by a Cubic Root |
|
| If you happen to multiply a square root by a cube root. | ואם יקרה לך לכפול שרש מרובע בשרש מעוקב |
|
נניח שרצית לכפול שרש מרובע מד' בשרש מעוקב מח' |
|
הנך צריך להשיב ד' לשרש מעוקב ויהיה לך שרש מעוקב מס"ד |
|
אח"כ תשיב ח' אל שרשי' ויהיה לך שרש ס"ד מרובע |
|
עתה תכפול שרש מרובע משרש מעוק' מס"ד |
| It can be expressed by the first way or the other. | כי יתכן לומר באופן האחד כמו באחר |
|
דהיינו ס"ד בס"ד ועולה ד' אלפי' וצ"ו |
|
ושרש מרובע מהשרש מעוקב או תאמ' השרש המעוקב משרש המרובע מד' אלפי' וצ"ו הוא הכפל האמור והוא ד' |
|
ג' בשרש מרובע מד' דהיינו שרש ט' בשרש ד' עולה שרש מל"ו שהוא ו' |
|
ג' בשרש מעוקב מח' שהוא שרש מעו' מכ"ז בשרש מעו' מח' עולה שרש מעו' מרי"ו והוא ו' |
|
שרש מרובע מד' בשרש מעוקב מח' עולה שרש מרובע משרש מעו' |
Multiplication of a Cube Root by a Root of a Root |
|
|
עוד אם יאמרו לך תכפול שרש מעוקב מח' בשרש שרש י"ו |
| Convert the number in the cube root to a root of a root, and the number in the root of the root to a cube root. | השב מספר השרש מעוקב לשרש השרש |
| Then, multiply them by each other, and the cube root of the root of the root, or the root of the root of the cube root of the result is the stated product. | ואח"כ תכפול זה בזה ושרש |
|
והנה הדמיון תכפול ח' באופן שרש השרש ואמור ח' מוכה בח' עושה ס"ד וס"ד מוכה בס"ד עולה |
|
אח"כ תכה י"ו באופן מעוקב ואמור י"ו מוכה בי"ו עולה רנ"ו ואח"כ אמור י"ו מוכה ברנ"ו שהוא המעוקב ועולה ד' אלפי' וצ"ו |
|
ואלו המספרי' תכפול זה בזה והם ד' אלפי' וצ"ו בד' אלפי' וצ"ו ועלו 16777216 |
|
והשרש מעוקב משרש השרש מהסך האמור או נאמ' שרש שרש מהשרש המעוקב מהסך האמור עולה בכפול שרש מעוקב מח' בשרש שרש י"ו העולה ד' במספר |
|
שרש מעוקב מח' מוכה בשרש משרש י"ו שרש מעו' משרש שרש או שרש משרש משרש שרש מעוקב מ 16777216 שהוא ד' |
Multiplication of a Number and a Root of a Number and a Root by a Number and a Root of a Number and a Root |
|
|
עוד אם רצית לכפול חצי ושרש מנוסף זינטו בלעז רביע אחד עם שרש י"ב בחצי אחד ושרש מנוסף רביע אחד עם שרש י"ב |
|
[25]אתה צריך ראשונה לכפול חצי בחצי ועושה רביע ושמרהו |
|
אח"כ תכפול חצי בשרש מנוסף רביע עם שרש י"ב ועולה שרש מנוסף א' מי"ו עם שרש ג' רביעי' |
|
אח"כ תכפול עוד בשתי וערב חצי בשרש מנוסף רביע עם שרש י"ב ועולה שרש מנוסף חלק מי"ו עם שרש ג' רביעי' |
|
ואלו שני הכפלי' השוים הם כאלו אמרת ב' בשרש מנוסף חלק מי"ו עם שרש ג' רביעי' שעולה שרש מנוסף רביע עם שרש י"ב ושמור |
|
אח"כ תכפול שרש מנוסף רביע עם שרש י"ב בשרש מנוסף רביע עם שרש י"ב שעולה רביע אחד ושרש מי"ב |
|
עתה תקבץ כל אלו הכפילות יחד ועולות חצי אחד ושרש מי"ב ויותר שרש מנוסף רביע עם שרש מי"ב |
|
וככה עולה הכפל האמור חצי ושרש מנוסף רביע עם שרש י"ב בחצי ושרש מנוסף רביע עם שרש מי"ב עולה חצי אחד ושרש מי"ב ושרש מנוסף רביע אחד עם שרש מי"ב |
|
| |
|
ודע כי כאשר תכפול המספר עם החלק מהמספר דהיינו חצי בשרש שני החלקי' הנוספים יחד דהיינו א' רביע נוסף עם שרש מי"ב אתה צריך להשיב החצי אל שרש ויהיה לך שרש [מא'] רביע |
|
ותכפול זה הרביע ברביע הנוסף עם שרש י"ב ועולה חלק אחד מי"ו |
|
אח"כ השב החצי האמור אל שרש משרש ויהיה לך שרש משרש מחלק מי"ו |
|
וזה החלק מי"ו תכפול בי"ב שהוא המספר הנקוב בשם שיש לו שרש ועולה ג' רביעים |
|
וקבץ זה הכפל יחד בקול דומה לאשר הוא כתוב לפנים ויהיה לך שרש נוסף עם שרש מג' רביעי' |
|
| |
| Then, perform the other similar multiplication crosswise. | ואח"כ תעשה בשתי וערב הכפל האחר הדומה לזה |
|
ויהיה לך הכפל הזה כפול אשר יהיה שנים בשרש הנוסף חלק מי"ו עם שרש ג' רביעי' |
|
ובגלל זה אתה צריך להשיב כמו כן זה השנים אל שרש ועולה ד' |
|
וזה הד' תכפול בחלק מי"ו שעולה חלק מד' |
|
אח"כ השב הב' האמור לשרש משרש ויהיה לך י"ו |
|
וזה הי"ו תכפול בג' רביעים ועולה י"ב |
|
וזה הכפל מקובץ יחד כפי הקול מהחלק מהכפל האמור ויהיה לך שרש מנוסף א' רביע עם שרש מי"ב |
|
| |
|
וככה עולה החלק מהכפל העשוי בשתי וערב |
| Now multiply the other multiplicands by themselves as said above, in order to complete the multiplication. | עתה תכפול החלקי' האחרים בעצמם כפי האמור למעלה להשלים הכפל |
| The reason you convert the number into a root, then into a root of the root: | והסבה שאתה תשיב המספר אל שרש ואח"כ אל שרש השרש |
| Because you multiply it by the root of the number that is added to a root of a number. | |
|
שהוא בשרש הנוסף רביע עם שרש מי"ב הוא כאלו כפלת ב' בשרש מנוסף חלק מי"ו עם שרש מג' רביעים |
|
אתה השיבות הב' אל שרש לכפלו עם שרש המספר הנוסף ואח"כ השיבות אותו לשרש משרש לכפלו |
|
|
|
חצי ושרש מנוסף רביע עם שרש י"ב בחצי ושרש מנוסף רביע עם שרש י"ב עולה |
|
| |
| Know that many other multiplications can fall into your hands. | [26]ודע כי כפלים אחרים רבים שונים מאלו יוכל להגיע לידך |
| They have no end, therefore it is impossible to write rules for all of them. | ואין סוף להם ולכן לא יתכן לכתוב כללים לכלם |
| Yet, from the aforesaid rules it is possible to understand and to present the rule, according to the aforesaid teaching, for every multiplication that comes, or that may come. | אבל מן הכללים האמורים יתכן להבין ולתת כלל כפי הלמוד האמור לכל כפל שיגיע ושיוכל לבא |
| Since, when multiplying the roots, one answers by saying the sum of a root of this and a root of that. | בהיות כי בכפול השרשי' תעשה תשובה בהאמר בסך שרש מכך ושרש מכך |
| Many times two or three or more types are summed, when a part of another part results from the multiplication, or when they cannot be summed together in one expression. | ופעמי' רבים ב' או ג' מינים ויותר נקבצים כאשר יעלו מהכפל הנעשה חלק אחר חלק וכאשר לא יתחברו יחד בקול אחד |
| Except for the roots that are equal and summed by doubling and duplicating: | לבד השרשי' אשר היו שוים ונתוספו באופן הכפל וההכפלה |
i.e. since when there are two equal roots, multiplying one of them by two yields the same as summing them together. 
|
דהיינו כי בהיות שני שרשי' שוים בהכפל אחד מהם בשנים עושה כך כמו בחברם יחד |
| and if there were three equal [roots] - multiplying one of them by three | ואם היו ג' שוים בהכפל אחד מהם בג' |
| וג"כ בהיות מינים יותר בהכפילם בכל כך מספר כמו שהם השרשי' השוים עושה כך כמו מחוברי' יחד | |
Addition and Subtraction of Roots |
|
| From here on it will be shown how similar and not similar roots can be summed together in one expression. | ומכאן ולהבא רצוני להראותך כיצד שרשים שוים ובלתי שוים יכולי' לחברם יחד בקול אחד |
| Since many are those roots that cannot be summed in one expression, | בהיות כי רבים הם אותם השרשי' אשר לא יתכן לחברם בקול אחד |
| and the nature of these roots, that cannot be summed together, is that when the numbers, by which the roots are denominated, are multiplied by each other, and that product does not have an expressible root, these are the roots that cannot be summed in one expression. | וטבע אותם השרשי' אשר לא יתכן לחברם יחד בקול אחד הוא זה כי בהכפל המספרי' אשר נקראי' שרשי' להם זה בזה ואותו הכפל אין לו שרש מדובר אלו השרשי' לא יתכן לחברם בקול אחד |
| Hence, these roots - which the multiplication of their numbers, by which they are denominated, by each other, yields a number that has an expressible root - can be summed in one expression, as will be demonstrated from here on. | א"כ אותם השרשי' אשר בהכפל מספריהם אשר נקראו שרשי' להם זה בזה יעשה מספר שיהיה לו שרש מדובר יתכן לחברם בקול אחד כמו שאראך מכאן ולהבא |
| The addition method of roots with roots and roots with numbers | בכאן יראה אופן חבור שרשי' עם שרשי' ושרשי' עם מספרי' או כאשר תרצה |
Addition of a Root with a Root |
|
|
|
נניח שרצית לחבר שרש ג' עם שרש י"ב |
|
|
הנך צריך לכפול שרש ג' בשרש י"ב ועושה שרש מל"ו ותקח שרש זה הל"ו שהוא ו' |
|
|
אח"כ תחבר מספרי השרשי' שהם ג' וי"ב יחד ויהיו ט"ו |
|
|
וזה הט"ו תוסיף על י"ב ששמרת ויהיו כ"ז ושרש מכ"ז הוא נקבץ שרש ג' עם שרש מי"ב |
| It is also possible to add the roots mentioned in this way: | עוד יתכן לחבר השרשי' הנזכרי' באופן זה |
|
|
תכה מספרי השרשי' זה בזה דהיינו ג' בי"ב ויעלה ל"ו וזה הל"ו תכה בד' ויהיה לך קמ"ד |
|
|
ותחברם עם מספרי השרשי' מקובצי' יחד דהינו על ט"ו ויהיה לך כ"ז ושרש זה הכ"ז הוא שני השרשי' מקובצים יחד כמו שאמרנו למעלה |
| For those that cannot be summed in one expression, the answer should be as they are, by expressing the one after the other: | ואותם אשר לא יתכן לחברם בקול אחד ראוי לתשובתם כמו מה שהם באמור האחד אחר האחר |
|
|
ונניח שרצית לחבר שרש ו' עם שרש ז' |
|
אתה צריך להשיב ולומר שרש מו' ושרש מז' עם שרש ז' ושרש ו' |
| If one wishes to answer in another way, the answer would be more difficult. | ואם רצונך להשיב לו באופן אחר יכבדו עליך יותר תשובותם |
| It is possible to answer in the aforesaid manner concerning the rule for those that are answered in one expression: | ותוכל לענות [27]להם בזה האופן האמור למעלה בכלל אותם אשר יענו בקול אחד |
|
|
והוא כי אתה צריך לכפול מספרי השרשי' זה בזה ועולה מ"ב וזה המ"ב תכה בד' ויהיה לך קס"ח |
|
|
ושרש קס"ח תחבר עם מספרי השרשי' הנקובי' בשם רצוני על שניהם שהוא י"ג ויהיה לך י"ג ושרש קס"ח ושרש זה הסך הוא שני השרשי' |
| Addition of a number and a root with a number and a root - adding the number to the number, then the roots to the roots, in the manner stated above. | ואם יזדמן לך לחבר מספר ושרש עם מספר ושרש אתה צריך לחבר המספרי' עם המספרי' ואח"כ השרשי' עם השרשי' באופן האמו' למעלה |
Subtraction of a Root from a Root |
|
| Know that as in the addition of the roots it was taught that summing two roots together is possible only for those, which when one of them is multiplied by the other, it yields a number that has an expressible root, likewise in the subtraction of the roots, it is possible to subtract the one from the other, so that the remainder will remain in one expression, only for those roots, which when one is multiplied by the other, it yields a number that has an expressible root. | ודע כי כמו שבחבור השרשי' נלמד כי לא יתכן החבור בקול אחד שני שרשי' יחד רק אותם אשר כשהוכה האחד באחר עושה מספר שיש לו שרש מדובר כמו כן בגרעון השרשי' לא יתכן לגרוע האחד מהאחר |
| As those that cannot be summed in one expression are stated as "a root of this plus a root of that", also those roots that cannot be subtracted in one expression can be answered as "a root of this minus a root of that". | וכמו שאותם אשר לא יתכן לחברם בקול אחד יאמר שרש מכך ושרש מכך כן ג"כ אותם השרשי' אשר לא יתכן להוציאם בקול אחד נוכל לענות שרש מכך פחות שרש מכך |
| This is by stating the greater first, minus the smaller, as I will show you in the example below: | וזה באמור הגדול תחלה פחות הקטון כמו שאראך לדמיון פה למטה |
|
נניח שרצית לגרוע שרש ג' משרש י"ב |
|
הנך צריך לפעול עם הכלל האמור בחבור בכמו שהוא להכות שרש ג' בשרש י"ב שעושה שרש ל"ו וקח שרשו המדובר שהוא ו' וכפלהו ויהיה י"ב ושמרם |
|
ואח"כ תחבר מספרי השרשי' יחד דהיינו ג' עם י"ב ועושה ט"ו |
|
|
וכמו שבחבור השרשי' יחובר הי"ב השמור כן בגרעון השרשי' צריך לגרוע מזה הט"ו הי"ב השמור וישאר ג' ושרש זה הג' הוא הנשאר מגרעון שרש ג' משרש י"ב |
| The other way that is done in the addition, is done also in the subtraction, | עוד באופן האחר שעושים בחבור כמו כן עושים במגרעת |
except that as the root of the product by 4 is added to the numbers of the roots that are summed together, 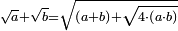
|
מלבד כי כמו שיחובר השרש מהכפל שהוכפל בד' על מספרי השרשי' שחוברו יחד |
so the aforesaid root is subtracted from the stated numbers that are summed together, and the root of the remainder is the remainder of the subtraction of a certain root from another root. 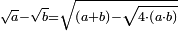
|
כן יגרע השרש האמור משני המספרי' האמורי' המחוברי' יחד ושרש הנשאר הוא השארית בגרעון שרש מה משרש אחר |
|
|
באופן זה ברצותך להוציא שרש ג' משרש י"ב תכה ג' בי"ב עושה ל"ו וזה הל"ו תכה בד' ועולה קמ"ד |
|
|
ועתה קבץ מספרי השרשי' אשר אתה בא להוציא האחד מן האחר שהוא ג' עם י"ב ועושה ט"ו |
|
|
ומזה הט"ו הוצא י"ב השמור וישאר ג' ושרש זה הג' הוא השארית הנשאר בהוציאנו שרש ג' משרש י"ב |
| Those roots that cannot be subtracted in one expression should be answered as they are, by stating the one, namely the greater, minus the other, namely the smaller: | ואותם השרשים אשר לא יתכן להוציאם בקול אחד ראוי לענות בהם כפי מה שהם באמור האחד רצוני הגדול פחות האחר רצוני הקטון |
|
|
ונניח שרצית להוציא שרש ו' משרש ז' |
|
ראוי אתה לענות [29]שישאר שרש ז' פחות שרש ו' |
| If one wishes to answer in another way, it is possible to answer according to the rule for those that are answered in one expression, although the answer will consist of the said combination: | ואם רצית לענות לו באופן אחר היית יכול |
|
|
בהיות כי אתה צריך לכפול שרש ו' בשרש ז' שעולה שרש מ"ב וזה המ"ב תכפול בב' שהוא בשרש ד' ויהיה לך שרש קס"ח |
|
|
ושרש זה הקס"ח תוציא משני מספרי השרשי' מחוברים יחד שהוא י"ג וישאר י"ג פחות שרש קס"ח ושרש זה השארית הוא מה שישאר מהוצאת שרש ו' משרש ז' |
Addition of a Number and a Root with a Number and a Root |
|
| After demonstrating the addition and subtraction of one root from another, it will be shown how to add or subtract a number and a root from a number and a root, or a number and a root from a number minus a root, and a root minus a number with a root minus a number, or from a root minus a number, in many ways shown from here on. | אחרי שהראיתיך לחבר ולהוציא שרש אחד מאחר רצוני להראותך כיצד נחבר או נוציא מספר ושרש ממספר ושרש או מספר ושרש ממספר פחות שרש ושרש פחות מספר עם |
|
|
ונניח שבקשת לחבר ד' ושרש י"ב עם ה' ושרש ג' |
| One should do as shown in the addition of roots, i.e. to sum the numbers with the numbers and the roots with the roots: | הנך צריך לעשות כאשר הראנו לך תחת חבור השרשים דהיינו שאתה צריך לחבר המספרי' עם המספרי' והשרשי' עם השרשי' |
|
|
ולכן תחבר ד' וה' ויהיו ט' ותשמרם |
|
אח"כ תחבר השרשי' באופן הכלל האמור בחבור |
|
|
דהיינו שרש ג' בשרש י"ב אשר עולה כ"ז |
|
|
אשר תחברהו אל המספר השמור שהוא ט' ויעלה הסך מאלו המספרי' והשרשי' מחוברי' יחד ט' ושרש כ"ז |
| בכאן יראה מספר ושרש עם מספר ושרש ובדברי' מה אחרים כאשר יראה בהמשך | |
Addition of a Number and a Root with a Root minus a Number |
|
|
|
ואם רצית לחבר ד' ושרש ג' עם שרש י"ב פחות ג' |
| It should be done with the rule stated for addition above, | צריך שתעשה עם הכלל האמור מהחבור למעלה |
| except that as for the addition of a number and a root with a root and a number the numbers should be summed together, | מלבד שכמו שבעבור חבור מספ' ושרש עם שרש ומספר צריך לחבר המספרי' יחד |
| so in order to add a root and a number with a root minus a number, one number should be subtracted from the other: | כמו כן לחבר שרש ומספר עם שרש פחות מספר צריך להוציא המספר האחד מהאחר |
|
|
הדמיון לזה לחבר ד' ושרש ג' עם שרש י"ב פחות ג' |
|
|
שצריך שנחבר שני השרשים יחד כאמור למעלה והנה חבורם יחד שרש מכ"ז ושמור |
|
|
ועתה הוצא ג' מד' וישאר א' וזה אתה מוציא בעבור כי הנך אומר ג' פחות |
|
|
א"כ ד' ושרש ג' עם שרש י"ב פחות ג' עולה שרש מכ"ז וא' יותר |
| ד' ושרש ג' בשרש י"ב פחות ג' עולה א' ושרש מכ"ז | |
|
|
עוד אם יאמר לך אדם חבר ד' עם שרש ג' עם שרש י"ב פחות ב' |
| One should subtract one root from the other, and one number from the other, since the name is denominated minus a root and minus a number, and the remainder will be the result of addition of a number minus a root with a root minus a number: | דע כי צריך אתה להוציא השרש האחד מהאחר והמספר האחד מהאחר בעבור כי נקוב בשם פחות שרש ופחות מספר |
Addition of a Number minus a Root with a Number minus a Root |
|
|
|
ומזה תקח המשל שיהיה ד' פחות שרש ג' עם שרש י"ב פחות ב' |
|
|
תוציא ב' מד' וישאר ב' פחות שרש ג' החלק האחד והאחר ישאר אחר זה שרש מי"ב |
|
|
עתה תוציא שרש ג' מהחלק האחר וישאר החלק האחד ב' והאחר הוא |
|
|
ולכן תוציא בדרך ההוצאה האמור לפנים שרש ג' משרש י"ב וישאר שרש ג' |
|
|
וזה השרש תחבר עם ב' האמור קודם ויהיה לך ב' ושרש ג' וככה עולה לחבר ד' פחות שרש ג' עם שרש י"ב פחות ב' |
| ד' פחות שרש ג' בשרש י"ב פחות ב' עולה ב' ושרש ג' | |
Subtraction of a Number minus a Root from a Number |
|
|
|
ואם רצית להוציא עשרה פחות שרש י"ב מי"ט |
|
|
הנך צריך לחבר השרש הפוחת מעשרה בחלק האחר דהיינו לשרש י"ב ויהיה לך י"ט ושרש י"ב |
|
|
ועתה צריך אתה להוציא עשרה מי"ט וישאר ט' |
|
|
א"כ להוציא עשרה פחות שרש י"ב מי"ט ישאר ט' ושרש י"ב |
Subtraction of a Number and a Root from a Number |
|
|
|
ואם רצית להוציא ח' ושרש נ' מי"ו |
|
|
הנך צריך להוציא ח' מי"ו וישאר ח' |
|
|
אח"כ הוציא שרש נ' מח' וישאר ח' פחות שרש נ' |
| מפני כי להוציא שרש מה ממספר מה לא יתכן לאמר יותר מדומה לזאת התשובה באמור המספר פחות השרש | |
|
|
א"כ להוציא ח' ושרש נ' מי"ו ישאר ח' פחות שרש נ' וכן נגמר המעשה |
Subtraction of a Number minus a Root from a Number |
|
|
|
עוד אם בקשת להוציא כ"ד פחות שרש מר"נ מעשרה |
|
|
הנך צריך לחבר שרש ר"נ על עשרה ויהיה לך עשרה ושרש ר"נ |
|
|
ועתה תוציא כ"ד מעשרה ושרש ר"נ יותר בזה האופן תוציא עשרה מכ"ד וישאר י"ד |
|
|
וזה הי"ד הוא פוחת א"כ ישאר האחר שרש ר"נ פחות י"ד ויצא לך כי בהוציאנו כ"ד פחות שרש ר"נ מעשרה ישאר שרש ר"נ פחות י"ד |
Subtraction of a Number minus a Root from a Number minus a Root |
|
|
|
עוד אם יאמר לך תוציא ו' פחות שרש ה' מי"ג פחות שרש כ' |
|
|
אתה צריך להוציא ו' מי"ג וישאר ז' |
|
|
ואח"כ תוציא שרש ה' משרש כ' אשר כפי הכלל מהוצאת שרש אחד משרש אחר אשר הראנו לך קודם ישאר שרש ה' |
|
|
ויגרע ג"כ השרש האמור א"כ להוציא ו' פחות שרש ה' מי"ג פחות שרש כ' ישאר ז' פחות שרש ה' |
| Many other additions, as well as other subtractions, which were not written, not seen, and not taught, can occur. | ודע כי הרבה חבורי' אחרים וכמו כן הוצאות אחרי' אפשר שיפלו אשר לא נכתבו גם לא נראו ולא נלמדו |
| Since the aforesaid rules of addition and subtraction of roots are enough for those stated above, and for any one that may occur, by observing each time what may be added or subtracted, as slightly seen in the stated rules. | מפני כי הכללי' האמורים למעלה מהחבור והמגרעת בשרשים הם מספיקים לאשר נאמרו למעלה ולכל אחד מאשר יוכלו להזדמן בהיותנו בכל פעם מתבוננים באשר אפשר להזדמן לחבר או להוציא כפי מה שהראית קצת בכללים האמורים |
Addition of Numerous Roots |
|
| If you wish to sum many types of roots together | [31]ואם רצית לחבר מינים רבים משרשים יחד |
|
|
נניח שרצונך לחבר שרש ג' עם שרש ו' ועם שרש י"ב ועם שרש כ"ד |
| וכמו כן שרשי' אחרים אשר יזדמנו לך | |
| The one should be multiplied by the other. | הנך צריך לכפול האחד באחר |
| If the product has no root - the product of one of the terms by one of the other terms should be investigated. | ואם אותו כפל לא יהיה לו שרש הנך צריך לחקור מכפול אחד מאותם החלקי' באחד מן החלקי' האחרי' |
| Then, what is discovered as possible to be summed, is summed in one expression | ולחבר בקול אחד אשר תמצא שאפשר לחברם |
| and what is not, is answered in the manner stated before for the roots that cannot be summed in one expression | ואשר לא תמצאם תענם באופן האמור קודם מהשרשי' אשר לא יתכן לחברם בקול אחד |
|
א"כ תכפול הראשון בשני דהינו שרש ג' בשרש ו' שעולה שרש י"ח |
|
וזה השרש אינו מדובר |
|
שני אלו השרשי' לא יתכן לחברם בקול אחד |
|
ולכן תנסה שרש ג' עם השרש השלישי שהוא שרש י"ב שעושה שרש ל"ו וזה השרש הוא מדבר והנה הוא ו' |
|
א"כ אלו שני השרשי' אפשר לחברם בקול אחד באופן האמור למעלה והנה הוא יהיה שרש מכ"ז |
|
ואם אלו השרשי' לא היה אפשר לחברם יחד בקול אחד אז היית מנסה ברביעי וכמו כן השני בשלישי וברביעי |
| וכן בנסות וכָפוֹל האחד באחר עד כלותך לנסות כל אחד מהם או מאחרים שיזדמנו | |
|
|
ודע כי השני אפשר לחבר בקול אחד עם הרביעי ויהיה שרש מנ"ד כאשר חוברו יחד |
| ויהיה לך כי אלו ד' השרשי' יהיו כאשר חוברו יחד בשני הסכי' רצוני הראשון בשלישי שהוא יהיה שרש מכ"ז והשני ברביעי שהוא יהיה שרש מנ"ד | |
|
| |
| ובזה האופן בעצמו ראוי שתבקש בכפול האחד על האחר ברצותך להוציא שרש אחד מב' שרשי' או ב' שרשי' מב' שרשים או ב' מג' וכדומה לזה בכל אופן מכמויות שרשים שיגיעו או שאפשר שיגיעו | |
|
|
א"כ לחבר שרש ג' ושרש ו' ושרש י"ב ושרש כ"ד יעלה שרש כ"ז ושרש מנ"ד |
Division of Roots |
|
| After you have seen the teaching of multiplication, addition and subtraction of roots, it remains for you to see the teaching of the division of roots, I mean one root by another root, a number by a root, a root by a number, a number and a root by a number, a number by a root and a number, a root by a number and a root, a number and a root by a number and a root, a root and a number by a number minus a root, and any manner that may occur | אחר אשר ראית התלמדות הכפל והחבור והמגרעת בשרשים נשאר לך לראות התלמדות החלוק בשרשים רצוני שרש אחד בשרש אחר או מספר בשרש או שרש במספר או מספר ושרש במספר או מספר בשרש ומספר או שרש במספר ושרש או מספר ושרש במספר ושרש או שרש ומספר במספר פחות שרש ובכל אופן שיוכל להגיע |
| Know that in the division of a certain root by another root you should divide the number of the first root by the number of the other, and the root of the quotient is the result of the division.
|
ודע כי בחלוק שרש מה בשרש אחר הנך צריך לחלוק המספר מהשרש האחד במספר מהשרש האחר ושרש מהמספר המגיע מן החלוקה הוא החלוק |
| Since the [root of the] quotient of one number by another number is the same as the expression of the root of the one from the expression of the root of the other | מפני כי כן הוא חלק מספר אחד ממספר אחר כקול שרש האחד מקול שרש האחר |
| Here is the example of this: | וזה הוא דמיונו |
|
נניח שרצית לחלק שרש ד' בשרש ט' |
|
הנך צריך לחלק ד' בט' שעולה ד' תשיעיות |
|
ושרש זה אלו הד' תשיעיות הוא החלוק הבא לחלוק שרש ד' בשרש ט' אשר זה הנגון או אמור הקול מד' תשיעיות המהות משרשו הוא ב' שלישיות |
|
אם כן לחלוק שרש ד' בשרש ט' עולה שרש מד' תשיעיות שהוא ב' שלישיות |
Division of a Number by a Root |
|
| If you wish to divide a number by a root: | [32]ואם רצית לחלק מספר בשרש |
|
ונניח שרצית לחלק ד' בשרש ט' |
|
הנך צריך ראשונה להשיב ד' אל שרש שיהיה לך שרש י"ו |
|
ועתה תחלק י"ו על ט' ויגיע א' וז' תשיעיות |
|
ושרש א' וז' תשיעיות הוא החלוק הבא בַחלוק שרש י"ו שהוא ד' האמור לחלק מספר בשרש ט' ושרש זה החלוק הוא א' ו |
|
א"כ לחלוק ד' בשרש ט' יגיע [שרש][33] א' וז' תשיעיות שהוא א' ושליש ונשלם |
Division of a Number by a Root and a Number |
|
| If you wish to divide a number [by] a root [and] a number: | ואם רצית לחלוק מספר ושרש במספר |
|
נניח שרצית לחלוק ח' בג' ושרש ד' |
|
הנך צריך לכפול ג' ושרש ד' בג' פחות שרש ד' ויעלה ה' |
|
א"כ לחלוק ה' בג' ושרש ד' יעלה לך ג' פחות שרש ד' |
| Since, for every number that is multiplied by another, when the product is divided by that number, the result is the other number by which it was multiplied.
|
מפני כי כל מספר שיוכה במספר אחר הכפל המגיע |
|
ולכן בחלוק ה' בג' ושרש ד' יגיע מזה ג' פחות שרש ד' |
|
ובחלוק ה' בג' פחות שרש ד' יגיע מזה החלק האחר שהוא שרש ג' ושרש ד' |
|
ולכן נאמר כי זה הה' יהיה המחלק |
|
ונשים זאת החלוקה בכלל הג' ונאמר אם מה' בחלקו בג' ושרש ד' יגיע ג' פחות שרש ד' כמה יגיע מח' אשר רצינו לחלקו |
|
דהינו אם מה' יגיע ג' פחות שרש ד' כמה ראוי להגיע מח' |
|
תכפול ג' פחות שרש ד' |
|
|
|
עתה נשאר לחלק שרש רנ"ו בה' דהינו בשרש כ"ה שיבא שרש עשרה וו' חלקי' מכ"ה |
|
| |
| Since in the division of roots by numbers, the number should be converted to roots, as it is converted in the division of numbers by roots. | מפני כי בחלוקת שרשי' במספרים צריך להשיב המספר אל שרשי' כמו שיושב בחלוקת מספרי' בשרשי' |
|
ולכן בחלוק |
Division of a Number and a Root by a Number |
|
| If you wish to divide a number and a root by a number: | ואם רצית לחלק מספר ושרש במספר ושרש |
|
נניח שרצית לחלק ה' ושרש י"ו בג' |
|
אתה צריך ראשונה לחלק המספר במספר דהיינו ה' בג' שיגיע א' וב' שלישים ושמרם |
|
אח"כ השב ג' המחלק אל שרשים ויהיה לך שרש ט' |
|
אח"כ חלק שרש מי"ו בשרש ט' ויגיע שרש מא' וז' תשיעיות |
|
דהיינו שרש מכמו שיגיע בחלוקת י"ו בט' |
|
א"כ לחלוק ה' ושרש י"ו בג' יעלה א' וב' שלישים ושרש א' וז' תשיעיות אשר זאת החלוקה בכלל תהיה ג' מפני כי שרש א' וז' תשיעיות הוא א' ושליש |
|
לחלוק ה' ושרש י"ו בג' יגיע א' וב' שלישי ושרש מא' וז' תשיעיו' שהם ג' שלמי' |
|
| |
|
והמספר הנחלק הוא ט' |
Division of a Number by a Number minus a Root |
|
| If you wish to divide a number by a number minus a root. | ואם רצית לחלק מספר במספר פחות שרש |
|
נניח שבקשת לחלק כ' בד' פחות שרש ט' |
| You should do it according to the aforesaid rule of the division of a number by a root and a number, except that when [you divide by a number and a root you should multiply] by a number minus a root, whereas in the division by a number minus a root, you should multiply by the inverse, i.e. by a number plus a root. | הנך צריך לעשות עם הכלל האמור לפנים מחלוקת מספר בשרש ומספר מלבד שאתה אם תכפול מספר ושרש במספר פחות שרש כן בחלוקת במספר פחות שרש צריך לכפול להפך דהיינו במספר ושרש |
| This is done in order that the divisor would be an integer. | אשר זה יעשה בסבת שהמחלק יהיה מספר שלם |
This teaching is found in the multiplication of roots, when a number plus a root is multiplied by the same number minus the same root [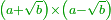 ], or a root plus a number by a root minus a number, when the numbers are equal to one another, meaning the subtractive to the additive, and the roots to each other [ ], or a root plus a number by a root minus a number, when the numbers are equal to one another, meaning the subtractive to the additive, and the roots to each other [ ], because the said products always yield integers ], because the said products always yield integers
|
וזה ההתלמדות [34]נמצא בכפילת השרשי' כאשר הזדמן שיכפל מספר ושרש בכך מספר פחות שרש כך |
|
ולכן תכה ד' פחות שרש ט' בד' ושרש ט' ועושה ז' שהוא המחלק לסבה האמורה קודם בחלוקת בעד מספר ושרש |
|
א"כ נאמר אנחנו אם מז' יגיע ד' ושרש ט' כמה ראוי להגיע מכ' שהוא אשר רצינו לחלק |
|
תכה ד' ושרש ט' בכ' ועולה פ' ושרש מג' אלפי' ת"ר |
|
וחלק בז' שיגיע י"א וג' שביעיו' ושרש ע"ג וכ"ג חלקי' ממ"ט |
|
א"כ לחלוק כ' בד' פחות שרש ט' יגיע י"א וג' שביעיו' ושרש ע"ג וכ"ג חלקים ממ"ט |
|
| |
| Know that when any of these divisions occur, or a division by a root minus a number, you should continue according to the said rule, to multiply always by the inverse of the denominator, as seen in the example. | דע כי כאשר יזדמן לך איזה מאלו החלוקי' או לחלוק בשרש פחות מספר תהיה ממשיך הענין בכלל האמור לכפול לעולם |
| Know also that when you divide a certain number [or] a root by a certain root and a number, such that the product of the added later by itself is greater than the product of the former by itself, then the addition, meaning the divisor, should be reversed, by placing the one whose product by itself yields more always first. | עוד דע כי כאשר יזדמן לך לחלוק מספר מה ושרש בשרש מה ומספר שאשר יחובר לאשר יהיה נקוב ראשונה בהכפל בעצמו יעשה יותר מאשר נקוב ראשונה בהכפל בעצמו צריך להפך הדיציאוני רצוני החלוק ולשים לעולם העושה יותר בהכפל בעצמו קודם |
|
והמשל בזה נניח שרצית לחלק י"ט בב' ושרש י"ו |
|
מן הכלל האמור הנך צריך לכפול ב' ושרש י"ו בב' פחות שרש י"ו |
|
אשר הכפל הזה לא יתכן וסבת זה היא כי שרש י"ו הוא יותר מב' והיותר לא נוכל להוציאו מהפחות |
|
וזה נוכל לראותו בפרהסיא כי בהכפל שרש מי"ו בעצמו עושה י"ו |
|
וב' בהכפל בעצמו עושה ד' |
|
א"כ שרש י"ו הוא יותר מב' שהוא שרש ד' |
|
וכמו שי"ו לא יתכן להוציאו מד' מפני שהוא יותר מד' כמו כן שרש י"ו לא יתכן להוציאו משרש ד' |
|
ולכן ברצותנו לחלק י"ט בב' ושרש י"ו או מהפכי' החלוק ונאמר בשרש י"ו וב' |
|
וזה נעשה אנחנו כדי לכפול שרש י"ו וב' בשרש י"ו פחות ב' וזה בטוב נוכל לעשותו ועולה י"ב |
|
א"כ נאמר עם כלל הג' אם מי"ב יגיע שרש י"ו פחות ב' כמה יגיע מי"ט |
|
תכפול שרש י"ו פחות ב' בי"ט ועולה שרש מה' אלפי' ותשע"ו פחות ל"ח |
|
ותחלקם בי"ב ויגיע שרש ממ' ותשיעית פחות שלשה ושתות |
|
א"כ לחלוק י"ט בב' ושרש י"ו יגיע שרש מ' ותשיעי' פחות ג' ושתות |
|
| |
| Knoe that when you have a number and a root [or] two equal roots, meaning that the root that you add to the number is as great as the number, or that there are two roots that are equal to one another, and one intend to divide by the sum of both, we cannot answer or deal with them by one of the said manners. | ודע כי אם יזדמן לך מספר אחד ושרש או אחד וב' שרשי' שוים רצוני כי השרש שתחבר עם המספר יהיה גדול כמו המספר או שיהיו ב' שרשים שוים האחד לאחר |
| The reason for this is that when we wish to multiply a number and a root by the same number minus the same root, as the root is equal to the number, the product will yield nothing. | והסבה למה היא זאת כי ברצותנו לכפול מספר ושרש במספר כמהו פחות שרש אחר כמהו בהיות השרש שוה [35]אל המספר לא יעלה דבר הכפל |
|
והנה המשל נניח שרצית לכפול ב' ושרש ד' בב' פחות שרש ד' |
|
ועושה מאומה נוּלַא בלעז |
|
מפני כי ככה הוא שרש ד' כמו שהוא ב' |
|
ולכן באמור שרש ד' פחות שוה לאומר מאומה |
|
ולכן אי אפשר לכפול ב' ושרש ד' בב' פחות שרש ד' |
|
וזה השרש אם ג"כ ב' שהוא כאומר ב' וב' בב' פחות ב' והינו ב' וב' פחות מאומה |
|
| |
| The same results from two equal roots. | וכדומה לזה יגיע מב' שרשי' שוים |
| Therefore, when you wish to divide by such divisors, one should sum the number with the root that is equal to it in one sum and to divide by that sum. | ולכן ברצותך לחלק בחלוקות כאלה צריך לחבר המספר עם השרש השוה לו בסך אחד ולחלק בסך ההוא |
| Also to sum the roots that are equal together in one sum and divide by that sum. | וכן צריך לחבר השרשי' הבלתי שוים יחד בסך אחד ולחלק בסך ההוא |
Division of a Number and a Root by a Number and a Root |
|
| If you wish to divide a number and a root by a number and a root. | ואם רצית לחלק מספר ושרש במספר ושרש |
|
|
|
תזכור שתהיה רוצה לכפול ה' ושרש ט' בה' פחות שרש ט' ועולה י"ו |
|
ואמור אם מי"ו יגיע לנו ה' פחות שרש ט' כמה יגיע מי"ט ושרש |
|
תכפול ה' ושרש ט' בי"ט ושרש כ"ה ועולה צ"ה ושרש תרכ"ה פחות שרש ג' אלפי' רמ"ט ופחות שרש רכ"ה |
|
ואלו תחלק בי"ו ויעלה ה' וט"ו חלקי' מי"ו ושרש ב' וקי"ג חלקי' מרנ"ו פחות שרש י"ב וקע"ז חלקי' מרנ"ו |
|
וזה החשבון השיבונו לשלמים והיו ג' וככה עולה בחלוק י"ט ושרש כ"ה בה' ושרש ט' |
|
| |
Division of a Number by Three Roots |
|
| If you wish to divide a certain number by three roots: | ואם רצית לחלק בג' שרשי' מספר מה |
|
ונניח שרצית לחלק ל"ו בשרש ד' ובשרש ט' ובשרש י"ו בדבר |
|
ואמור מאלו השרשי' כאלו הם מדוברים אתה צריך לכפול שרש ד' שעולה שרש קמ"ד פחות ג' מספרי' |
|
א"כ לחלוק שרש קמ"ד פחות ג' בשרש ד' ושרש ט' ובשרש י"ו יגיע לך שרש ד' ושרש ט' פחות שרש י"ו כפי מה שהראית לפנים בכלל חלוק מספר בשרש ומספר |
|
ולכן נאמר כי זה השרש פחות מספר רצוני שרש מקמ"ד פחות ג' אשר הוא כפל ממה שנאמ' קודם הנה הוא יהיה מכאן ולהבא מחלק |
|
ונאמר בכלל הג' אם שרש קמ"ד פחות ג' נותן שרש ד' ושרש ט' פחות שרש י"ו מה יתן ל"ו אשר אמרנו למעלה לחלוק בג' השרשי' |
|
תכפול שרש ד' ושרש ט' פחות שרש י"ו בל"ו שעולה שרש מה' אלפי' וקפ"ד ושרש מי"א אלפי' תרי"ד פחות שרש כ' אלפי' ותשל"ו |
|
וזה תחלק בשרש קמ"ד פחות ג' אשר זה המחלק |
|
| |
|
נוכל להשיבו אל מספר מדבר מפני כי קמ"ד יש לו שרש מדבר |
|
ולכן נאמ' עוד באופן חלוקת הקודם בשרש פחות מספר תכפול שרש מקמ"ד פחות ג' בשרש קמ"ד וג' יותר עולה קל"ה |
|
ונאמר מראש שזה הכפל הוא המחלק |
|
ונשוב אל הכלל מהג' ונאמר אם מקל"ה יגיע קמ"ד וג' יותר כמה יגיע משרש ה' אלפי' וקפ"ד ומשרש י"א אלפי' תרס"ד פחות שרש כ' אלפי' תשל"ו |
|
[36]תכפול שרש קמ"ד וג' יותר בשרש ה' אלפי' וקפ"ד ושרש מי"א אלפי' תרס"ד פחות שרש מכ' אלפי' תשל"ו ועולה שרש מתשמ"ו אלפי' ותצ"ו ושרש מאלף ותרע"ט אלפי' ותרי"ו ושרש מ"ו אלפי' ותרנ"ו ושרש ק"ד אלפי' ותתקע"ו פחות שרש מ 4895892 ופחות שרש מ 426681 |
|
וזה הכפל תחלק בקל"ה מושב לשרש שעולה שרש מ' וכ"ד חלקי' מכ"ה ושרש צ"ב וד' חלקי' מכ"ה ושרש ב' וי"ד חלקי' מכ"ה ושרש ה' וי"ט חלקי' מכ"ה פחות שרש קס"ג וכ"א חלקי' מכ"ה ופחות שרש עשרה וו' חלקי' מכ"ה |
|
וככה עולה |
|
| |
Division of a Number by Four Roots |
|
| If you wish to divide a certain number by four roots: | ואם רצית לחלק מספר מה בד' שרשי' |
|
ונניח שרצית לחלק ע' בשרש ד' ובשרש ט' ובשרש י"ו ובשרש כ"ה מחוברי' כלם יחד באופן כאלו היו השרשי' האלו בלתי מדוברי' |
| You should create one product from these four roots, always placing the greater root first, as you have seen, this way: | הנך צריך לעשות כפל אחד מאלו הד' שרשי' בשומך לעולם הגדול מהשרשי' קודם כפי מה שהראית באופן זה |
|
כאמור שרש כ"ה ושרש י"ו ושרש ט' ושרש ד' בשרש כ"ה ושרש י"ו פחות שרש ט' ופחות שרש ד' ועולה שרש כ"ח ושרש אלף ת"ר פחות שרש קמ"ד |
|
| |
|
ונאמר כי בחלוק כ"ח ושרש אלף ת"ר פחות שרש קמ"ד בשרש כ"ה ובשרש י"ו ובשרש ט' ובשרש ד' יעלה שרש כ"ה ושרש י"ו פחות שרש ט' ופחות שרש ד' |
|
אשאל א"כ כמה יעלה מע' |
|
הנך צריך לכפול כפי הכלל מהג' ע' בשרש כ"ה ושרש י"ו פחות שרש ט' ופחות שרש ד' שעולה שרש מקכ"ב אלפי' ות"ק ושרש מע"ח אלפי' ות' פחות שרש מ"ד אלפי' וק' ופחות שרש מי"ט אלפי' ות"ר |
|
| |
|
וזה הכפל הנך צריך לחלק בכ"ח ושרש אלף ות"ר פחות שרש קמ"ד |
|
וזה החלוק הוא ג' [קשרים][37] קלאפי בלעז ולכן תצטרך להמשך כפי הכלל מהחלוק בג' שרשים ולעשות שרש כ"ח ושרש אלף ת"ר פחות שרש קמ"ד בכ"ח ושרש אלף ת"ר ויותר שרש מקמ"ד שעולה אלפיים ור"מ ושרש מ 0067105 |
|
| |
|
וזה הכפל כאשר נחלק בכ"ח ושרש אלף ת"ר פחות שרש קמ"ד יעלה כ"ח ושרש אלף ת"ר ויותר שרש קמ"ד |
|
אשאל כמה יעלה משרש קכ"ב אלפי' ות"ק ושרש ע"ח אלפי' ות' פחות שרש מ"ד אלפי' וק' ופחות שרש י"ט אלפי' ות"ר |
|
הנך צריך לכפול כפי הכלל מהג' כ"ח ושרש אלף ת"ר ועוד שרש קמ"ד בשרש קכ"ב אלפי' ות"ק ושרש ע"ח אלפי' ות' פחות שרש ממ"ד אלפי' וק' ופחות שרש מי"ט אלפי' ת"ר שעולה שרש מ 00004069 ושרש |
|
| |
|
וזה הכפל תחלק באלפיים ר"מ ושרש מ 0067105 אשר זה המחלק הוא [38]מב' קשרי' קלאפי בלעז שהם שוים |
|
ואם היה אחד מהם גדול מהאחר היינו מתעסקי' בזה באופן החלוק |
|
אבל אי אפשר עתה להמשך כפי מה שנאמר קודם מפני כי צריך לחבר החלקי' יחד בקול אחד כאשר הם בלתי שוים |
|
לכן תחבר אלפיים ור"מ עם שרש מ 0067105 שהוא אלפיים ור"מ ועולה ד' אלפי' ות"פ וככה הוא המחלק האמור |
|
אם כן תחלק הכפל האמור וככה יעלה לחלוק ע' בשרש ד' ובשרש ט' ובשרש י"ו ובשרש כ"ה |
|
ואלו שרשי החלוקה כאשר הושבו למספר מדובר יהיה סכומם ה' מספרים שלמים |
|
| |
| It is also possible to divide it by the stated roots or by any other four roots in a different way that seems more difficult at first than the way you saw, but it is easier later in the procedure, and it is required when you wish to divide by more roots. This is the way: | עוד אפשר לחלקו בשרשים האמורי' או בד' שרשי' אחרים שיזדמנו באופן אחר הנראה יותר חמור בהתחלה מן האופן אשר הראית אבל הוא יותר נקל בהמשך הפעל ג"כ הוא מוצרך ברצותך לחלק ביותר שרשים וזה הוא זה האופן |
|
נניח שרצית לחלק ע' בשרש ד' ובשרש ט' ובשרש י"ו ובשרש כ"ה מחוברי' באופן כאלו היו שרשי' בלתי מדברי' |
| Sum up the two smaller together and the two greater together in a way they can be summed, if any of them cannot be combined into one expression. | תחבר שני הקטני' יחד ושני הגדולים יחד באופן שאפשר לחברם אם לא יתכן חבורם איזה מהם יחד בקול אחד |
| Assuming that when one is multiplied by the other the result is an inexpressible root: | בהניח כי כשיכפל האחד באחר יעשה שרש בלתי מדבר |
|
ולכן בחבר שרש ד' בשרש ט' באופן האמור עושה שרש מחבור שרש קמ"ד עם י"ג |
|
אח"כ תחבר שרש י"ו עם שרש כ"ה ועושה שרש מחבור שרש אלף ת"ר עם מ"א בחבר אליו באופן האמור קודם דהיינו באופן השני מאלו אשר אי אפשר לחברם בקול אחד |
| Place the two greater roots before the two smaller as they are summed. | אח"כ תשים שני השרשי' הגדולי' קודם שני הקטני' כפי מה שהם מחוברי' |
| Multiply them by the two greater addends minus the two smaller addends. | ותכפלם בשני הגדולי' מחוברי' פחות שני הקטני' מחוברי' באלו |
|
באמור שרש מחבור אלף ת"ר עם מ"א ושרש מחבור שרש קמ"ד עם י"ג בשרש מחבור שרש אלף ת"ר עם ועולה כ"ח ושרש אלף ת"ר פחות שרש קמ"ד |
|
| |
|
עתה תשוב אל הכלל מהג' ואמור אם מזה הכפל רצוני מכ"ח ושרש אלף ת"ר פחות שרש קמ"ד יגיע שרש מחבור שרש מאלף ת"ר עם מ"א פחות שרש מחבור קמ"ד עם י"ג כמה יעלה מע' |
|
תכפול שרש מחבור אלף ת"ר עם מ"א פחות שרש מחבור שרש קמ"ד עם י"ג בע' שעולה שרש מחבור שרש מ 00000061483 עם שרש מ 009002 פחות שרש מחבור שרש מ 0000447543 עם 00736 |
|
| |
|
וזה תחלק [39]על כ"ח ושרש אלף ת"ר פחות שרש קמ"ד אשר זה המחלק הוא מג' קשרים |
| It should be continued according to the rule of division by three as we showed above. | וצריך להמשך כפי כלל החלוק בג' כפי מה שהראינו קודם |
| You can also divide by these three terms according to the way we introduced above in this chapter. | וג"כ תוכל לחלק באלו ג' קשרים כפי האופן אשר המשכנו למעלה בזה הכלל |
|
רצוני בחבר כ"ח שהוא שרש מתשפ"ד עם שרש אלף ת"ר שעולה שרש מחבור מ 0067105 עם 4832 |
|
אשר זה השרש א' סכום יש לו שרש אחד פחות דהיינו שרש קמ"ד |
|
ולכן תכפול שרש מחבור שרש מ 0067105 עם שרש מ 4832 פחות שרש קמ"ד בשרש מחבור שרש מ 0067105 עם 4832 ויותר שרש מקמ"ד שעולה 0422 ויותר שרש מ 0067105 |
|
| |
|
עתה צריך אתה |
|
תכפול שרש מחבור שרש מ 0067105 עם שרש אלפיים ושפ"ד ויותר שרש קמ"ד בשרש מחבור שרש מ 00000061483 עם 009002 פחות שרש מחבור שעולה שרש מחבור שרש מ 000000006121657291 עם שרש מחבור שרש מ 000000652004415202 |
|
| |
|
וזה הכפל תחלק ב 0422 ויותר שרש מ 0067105 אשר המחלק הוא מב' קשרים |
| The procedure should continue according to the rule of division of two roots, if the terms are not equal. | וצריך להמשיך הענין כפי הכלל מהחלוק מב' שרשי' אם הקשרי' היו בלתי שוים |
|
אבל מפני שהם שוים רצוני ששרש מ 0067105 הוא אלפיים ור"מ אנחנו נחלק בכפלו שהוא ד' אלפים ות"פ |
|
שיגיע ממנו שרש מחבור שרש מתע"ח ואלפיים קי"ב חלקי' מד' אלפי' צ"ו עם שרש מחבור תק"ב וג' אלפי' וש"ג מד' אלפי' צ"ו |
|
| |
|
ובסכום יהיה שרש מצ"א |
|
ויותר שרש מחבור שרש א' וד' אלפי' וד' חלקי' מד' אלפי' וצ"ו עם א' וקי"ג חלקי' מרנ"ו שעולים לסך שרש ב' ורי"ז חלקי' מרנ"ו אשר זה השרש הוא א' וי"א חלקי' מי"ו |
|
ובחברם עם השרש הראשון שהוא ט' וט' חלקי' מי"ו ועולה י"א ורביע |
|
| |
|
פחות שרש מחבור שרש מ"ג ורע"ב חלקי' מתצ"ו עם שרש מחבור שרש נ' ואלפיים רכ"ה חלקי' מד' אלפי' וצ"ו |
|
| |
|
[40]פחות שרש מחבור שרש 0 ותשכ"ט חלקי' מד' אלפי' וצ"ו עם 0 וקי"ז חלקי' מרנ"ו |
|
וחבורו עם השרש הראשון מזה הפחת שהוא ה' |
|
אם כן ישאר ה' וככה יעלה לחלוק ע' בשרש ד' ובשרש ט' ובשרש י"ו ובשרש כ"ה |
|
| |
|
לחלק ע' בשרש ד' ובשרש ט' ובשרש י"ו ובשרש כ"ה מחוברי' יחד |
|
יעלה שרש מחבור שרש מתע"ח ואלפיים קי"ב חלקי' מד' אלפי' וצ"ו עם שרש מחבור שרש מתק"ב וג' אלפי' ול"ג חלקי' מד' אלפי' וצ"ו |
|
ויותר שרש מחבור שרש א' וד' אלפי' וד' חלקי' מד' אלפי' וצ"ו עם א' וקי"ג חלקי' מרנ"ו |
|
פחות שרש מחבור מ"ג ורע"ב חלקי' מד' אלפי' וצ"ו עם שרש מחבור שרש נ' וב' אלפי' רכ"ו חלקי' מד' אלפים וצ"ו |
|
ופחות שרש מחבור שרש 0 ותשכ"ט חלקי' מד' אלפי' וצ"ו עם 0 וקי"ז חלקי' מרנ"ו |
|
| |
|
ומושב הכל למספר מדובר יעלה אל סך מה שיעלה מן החלוקה שהוא חמשה |
| Know that you may encounter many other types of division; they are endless. | ודע כי מיני' רבים אחרי' מהחלוק אפשר שיזדמנו לך והם בלי תכלית |
| But, from the teachings we have presented above, you can deduce a general rule for every multiplication, division, subtraction and addition you may encounter. | אבל מן הלמודי' אשר הגדנו למעלה תוכל לתת כלל לכלם לכפל ולחלוק ולמגרעת ולחבור שיוכלו להזדמן לך |
Algebra |
|
The Six Canonical Equations |
|
| There are six chapters in the book al-Jīblī al-Mūqabāla: three of them are simple and the others are compound; the third of the three simple can be returned to the first. | בספר אלג'יבְלֵי אמוגאבאלא יש בו ששה פרקים ומהם שלשה פשוטי' והאחרים הם מורכבי' והשלישי מהג' הפשוטי' אפשר להשיבו אל הראשון |
| One can observe these chapters and create many other species that can be returned to this nature or to the similarity of the mentioned chapters, as you can see in the example of the amendments that are set from here on. | ועל אלו הפרקי' אפשר להתבונן ולעשות מיני' רבים אחרים אשר בעבור זה ישובו אל הטבע או אל דמיון הפרקי' האמורי' כפי אשר תראה במשל בתקוני' אשר נניח מכאן ולהבא |
| By these chapters, with the amendments, it is possible to reach through demonstration to profound concealed subtle calculations, either of arithmetic or of geometry. | אשר עם אלו הפרקי' עם התקוני' אפשר להגיע בבאור אל חשבונו' עמוקי' ונסתרי' ודקים בין מהאריסמיטיקא ובין מהגימטריא |
| The chapters and their nature are written below: | אלו הכתובי' תחת זה הם הפרקי' וטבעם |
|
הפרק הראשון יבא דבר שוה למספר |
|
הפרק השני יבא צינסו שוה למספר |
|
הפרק השלישי יבא דבר שוה לצינסו |
|
הפרק הרביעי יבא צינסו ודבר שוה למספר |
|
הפרק החמישי יבא צינסו ומספר שוה לדבר |
|
הפרק הששי יבא צינסו ומספר שוה לצינסו |
|
[41]הטבע מן הפרק הראשון הוא זה כאשר הדברי' יהיו שוים אל המספר |
|
צריך לחלק המספר בכמות הדברים והעולה מהן שוה הדבר |
|
נניח המשל ונאמ' כי שלשה דברי' יהיו שוים לי"ב |
|
חלק המספר שהוא י"ב בכמויות הדברי' שהם ג' ויעלה מהם ד' וככה שוה הדבר |
|
אם כן אם הדבר הוא ד' ג' דברי' היטב הם שוים אל י"ב |
|
הטבע מן הפרק השני הוא זה כאשר הצינסי יהיו שוים אל המספר |
|
צריך לחלק המספר בכמויות הצינסי והעולה מזה ככה שוה |
|
והדבר הוא השרש מאשר יגיע מפני כי הדבר הוא שרש מהצינסו |
|
נניח כי ב' צינסי יהיו שוים אל ל"ב |
|
תחלק המספר שהוא ל"ב בכמויות הצינסי שהם ב' ויהיה המגיע י"ו וככה שוה הצינסו |
|
והדבר הוא שרשו דהיינו שרש מי"ו שהוא ד' |
|
ולכן אם הצינסו הוא י"ו ב' צינסי היטב יהיו שוים לל"ב |
|
הטבע מהפרק השלישי הוא זה כאשר הדברי' הם שוים לצינסי |
|
צריך לחלק כמויות הדברי' בכמויות הצינסי והעולה מזה הוא מספר וככה שוה הדבר |
|
נניח כי ב' צינצי יהיו שוים אל ו' דברים |
|
תחלק כמויות הדברי' שהם ו' בכמויות הצינסי שהם ב' ויהיה העולה ג' וככה שוה הדבר |
|
וזה הדבר בהיותו ג' הצינסו יהיה ט' ואם הצינסו הוא ט' א"כ ב' צינסי יהיו י"ח |
|
ובהיות הדבר ג' ו' דברי' יפה הם שוים י"ח ולכן ב' צינצי יהיו היטב שוים לו' דברי' |
|
ודע כי זה הפרק השלישי אפשר להשיבו אל הראשון כפי האמור למעלה אֵיסְקִיסאנְדּוֹ האַדֵּיקְוואצִיאוֹנֵי הוא התקון בדבר כמו שתראה בהמשך הספר האמור |
|
הטבע מהפרק הרביעי שהוא הראשון מהמורכבי' בעבור כי אחד או כמות אחד יזדמן שיושם שוה לשני כמויות אחרים מתחלפים הוא זה כאשר הצינסי והדברי' יהיו שוים למספרי' |
|
צריך לחלק כל הדיקוואציאוני בכמויות הצינסי |
|
ואח"כ לחלק כמויות הדברי' לשני חלקי' שוים |
|
ואחד מאותם החלקי' שהוא חצים תכה בעצמו |
|
והוסיפם על כמויות המספר |
|
ושרש זה הסכום פחות החצי האחר מן הדברי' הוא הדבר |
|
והנה המשל נניח כי ב' צינסי וכ' דברי' יהיו שוים אל |
|
הנך צריך עתה לחלק כל הדיקוואציאוני בכמויות הצינסי |
|
ויהיה לך צינסו אחד וי' דברי' שוים לל"ט |
|
אח"כ תחלק כמויות הדברי' לחצי רצוני על ב' ויהיה כל אחד מהחלקי' ה' |
|
וזה הה' תכפול בעצמו ויהיה לנו כ"ה |
|
והוסיף אלו הכ"ה על המספר שהוא ל"ט ויהיה לך ס"ד |
|
ושרש זה הסך רצוני שרש ס"ד פחות החצי האחר מהדברי' הוא הדבר דהיינו פחות ה' וזה הדבר בהרצות במספר ידוע [42]יהיה ג' |
|
והסבה היא זאת כי שרש ס"ד הוא ח' |
|
ומזה הח' נוציא החצי מכמויות הדברי' שהוא ה' וישאר ג' א"כ הדבר הוא ג' |
|
| |
|
והצינסו הוא הכאתו בעצמו שהוא ט' |
|
א"כ בהיות הצינסו האחד ט' וי' דברי' כשישוה הדבר ג' יהיו ל' וכשיחוברו יחד יהיו היטב שוים אל ל"ט |
|
הטבע מהפרק החמישי רצוני השני מהמורכבי' הוא זה כאשר הצינסי והמספר יהיו שוים למספר |
|
צריך לחלק כל האדיקוואציאוני בכמויות הצינצי |
|
ואח"כ לחלק הדברי' לשנים |
|
ואחד מאותם החצאים רצוני הכמות מאחד מאותם החלקי' תכפול בעצמו |
|
ומאותו הכפל תוציא המספר |
|
ושרש הנשאר תוסיף על החצי האחר מכמות הדברי' וככה יהיה שוה הדבר |
|
או תוציא שרש הנשאר מהחצי האחר מכמות הדברי' וככה יעלה שווי הדבר |
| ודע כי חשבונות מה תצטרך להשיב שיהיה הדבר באופן הראשון רצוני שיהיה החצי מכמות הדברי' ויותר שרש מהנשאר | |
| וחשבונות אחרי' מה באופן השני שהוא החצי מכמות הדברי' פחות שרש הנשאר | |
| ויש אשר אפשר לענות בם בשני האופני' | |
|
והנה המשל נניח כי ג' צינסי וס"ג מספרי' יהיו שוים לל' דברי' |
|
הנך צריך לחלק ראשונה כל האדיקוואציאוני בכמות הצינסי שהוא ג' |
|
ויהיה לך א' צינסו וכ"א מספרי' שוים לי' דברי' |
|
אח"כ תחלק כמות הדברי' לחצי ויהיה לך כל חלק ה' |
|
וזה הה' תכפול בעצמו ועולה כ"ה |
|
ומהם תוציא המספר שהוא כ"א וישאר ד' |
|
ושרש ד' תוסיף על החצי האחר מהדברי' או תגרע ויגיע לך כי הדבר יהיה ה' ושרש ד' או ה' פחות שרש ד' |
|
| |
| ופעמי' מה תוכל להשיב שהדבר הוא כפי האופן הראשון ולא כשני ופעמי' מה כפי השני ולא כראשון כמו שתראה מכאן ולהבא בחשבונות מה יושמו לזה הקפיטולו | |
|
|
ואם תשיב היות הדבר כפי האופן הראשון שהוא ה' ושרש ד' שהוא ב' יהיה לך היות הדבר ז' |
|
|
ואם הדבר הוא ז' י' דברי' יהיו ע' |
|
|
והצינסו יהיה מ"ט בהיות הדבר ז' |
|
|
א"כ א' צינסו עם כ"א יותר יגיע היטב להיות שוה לי' דברים |
|
|
ואם תענה היות הדבר כפי האופן השני שהוא ה' פחות שרש ד' אשר זה השרש הוא ב' ישאר להיות הדבר ג' |
|
|
וי' הדברי' יהיו ל' |
|
|
והצינסו יהיה ט' בהיות הדבר ג' |
| א"כ א' צינסו עם כ"א יותר יהיו היטב שוים אל י' דברים בשני האופני' | |
| אבל הכרח מענה האופן הראשון עם של השני אי אפשר לראותו במשל הפרק לבד למה יתן להיות הדבר באופן | |
|
הטבע מהפרק הששי שהוא השלישי המורכב הוא זה כאשר הדברי' והמספרי' יהיו שוים אל הצינסי |
|
[43]צריך לחלק [44]כל האדיקוואציאוני בכמות הצינסי |
|
ואח"כ לחלק הדברי' לשנים |
|
ואחד מאלו החצאי' רצוני כמותו תכפול בעצמו |
|
והכפל הזה תוסיף על המספרי' |
|
ושרש כל הסכום ויותר כמות מחצית הדברי' הוא הדבר |
|
והנה המשל נניח כי שלשה דברי' וד' דרמי רצוני ד' מספרי' יהיו שוים אל א' צינסו |
|
הנך צריך לחלק כל האדיקוואציאוני בכמות הצינסי שהוא א' ויבא |
|
ולכן תחלק כמות הדברי' לחצי ויהיה לך א' וחצי |
|
תכפלהו על עצמו ויגיע ב' ורביע |
|
וזה תוסיף על המספר ויהיה לך ו' ורביע |
|
ושרש ו' ורביע ויותר החצי האחר מכמות הדברי' הוא הדבר |
|
ושרש ו' ורביע הוא ב' וחצי |
|
ובחברו על מחצית כמות הדברי' שהוא א' וחצי עושה ד' |
|
| |
|
|
א"כ אם הדבר הוא ד' והצינסו יהיה הכאתו בעצמו והוא י"ו |
|
|
ובהיות הדבר ד' ג' דברי' יהיו י"ב |
|
|
א"כ ג' דברי' וד' יותר היטב יהיו שוים לא' צינסו שהוא י"ו |
| בכאן יראה התחכמות האדיקוואציאוני הם השאלות לפי דעתי | |
| דע כי לעולם אם יזדמן לך איזו שאלה מורכבת שתחלק לעולם כל השאלה בכמו שהוא כמות הצינסו ולהשיבה לעולם לא' צינסו כאשר הראית בשאלות מהג' פרקי' המורכבי' האמורי' למעלה | |
| וכן בדומה לזה תמשיך הענין בכל אחת מהשאלות המורכבות לחלק לעולם כל השאלה בכמות אשר באחדותו יש לו מהות גדול מאחדות מה מכמויות שיהיו | |
| ואח"כ תמשיך טבע הפרק כפי מה שהראית ושעתיד להראות מכאן ולהבא | |
Geometric Illustrations of the Three Compound Equations |
|
| בכאן יראה צורת הג' פרקים המורכבים | |
| עוד רצוני להראותך טבע אלו הג' פרקי' המורכבים בצורת כל אחד לבדו | |
|
|
איך א' צינסו וי' דברי' יבא מופת שהם שוים אל ל"ט |
|
בהיות כי הדבר אשר נקבנו בשם הוא שרש הצינסו ולכן יהיה הצינסו שטח אחד מרובע נצב הזויות שוה הצלעות |
|
|
|
|
ולכן נצייר צורת זה מרובע אחד שוה הצלעות והזיות נצבות ונאמר כי זה המרובע הוא הצינסו והוא השטח שעליו א"ב |
|
ומפני כי הדבר הוא שרש הצינסו יהיה צלעו' למרובע האמור |
|
ובהיות כי נוסף על הצינסו י' דברים אנו נחלק אלו הי' דברים בד' חלקים ויגיע לכל חלק ב' דברים וחצי |
|
ובהיות כי הדבר הוא צלעות הצינסו אנחנו נדביק כל אחד מאלו הד' חלקי' [45]אל הצינסו וכל חלק לבדו לצלעו מהצינסו |
|
ויהיו לנו ד' שטחי' כל אחד מהם יהיה רחבו ב' וחצי וארכו כאורך צלעות הצינסו ושטח כל אחד מהם עליו ג"ד |
|
ולכל אחד מזויות הצינסו יהיה עליו מרובע אחד שוה הצלעות והזיות נצבות ויהיה רחבו בצלעותיו כרחב הדברי' שהוא ב' וחצי |
|
וכל אלו הד' שטחי' שוי הצלעות והזויות נצבות כאמור והם באורך וברחב שוים לרחב הדברי' ושטחיהם יחד |
|
וצלעותיהם ו"ז והוא ב' וחצי |
|
וא"כ יהיה לנו עתה מרובע אחד המחזיק הצינסו ועשרת הדברי' |
|
וג"כ אלו הד' |
|
ושטח הצינסו יהיה עם שטח הדברי' ל"ט |
|
ומחובר עם כ"ה שהוא שטח הד' מרובעי' השוי הצלעות עושה ס"ד |
|
א"כ יהיה לנו מרובע אחד אשר יחזיק כל אלו השטחי' ושטחו יהיה ס"ד עליו ח"ט ויהיה שוה הצלעות והד' זויות |
|
ולכן צלעו יהיה שרש ס"ד |
|
וצלע הסינסו יהיה ה' פחות |
|
מפני כי הדברי' אשר ממעל עם אשר מתחת או ג"כ המרובעי' מהזויות יש להם ממרחב ב' וחצי |
|
והנה כי ב' וחצי ממעל וב' וחצי מתחת מחוברי' יחד עושה ה' |
|
אם כן יהיה צלע הצינסו ה' פחות מצלע המרובע המחזיק כל אלו השטחי' |
|
וכמו שראית זה מפני רוחב הדברי' ממעל ומתחת וכדומה לזה תוכל לראות ברוחב הדברי' מהצלעות |
|
מפני כי כל צלע שוה רצוני צלעות הצינסו הם שוים זה לזה |
|
וצלעות המרובע הגדול שוים זה לזה |
|
א"כ יהיה הדבר שהוא צלע הצינסו שהוא שרש הצינסו שרש ס"ד פחות ה' |
|
וזה השרש במספר מדובר הוא ג' |
|
ואם הדבר שהוא שרש הצינסו יהיה ג' הצינסו יהיה הכאתו בעצמו שהוא ט' וזה הט' הוא שטח הצינסו |
| I want to show you this in a different way: | עוד רצוני להראותך זה באופן אחר |
|
באמרנו בדומה לזה שצינסו אחד ועשרה דברים או אמור עשרה שרשיו הם שוים לל"ט |
|
|
|
|
אנחנו נצייר עתה מרובע אחד שוה הצלעות מד' זויות בעבור הצינסו ועל השטח רשמנו ב"ג |
|
ועשרת הדברים אנו מחלקים לב' חלקים ויהיה לנו כי כל חלק יהיה ה' שרשים מהצינסו או אמור ה' דברים |
|
אשר אנחנו נדביק כל חלק אל צלע הצינסו כפי אשר תראה מצוייר פה בזאת הצורה |
|
ויהיה לנו שני אלו החלקים מדובקים לשני צדדי הצינסו אשר אלו הצדדים מחזיקים זוית אחד ושטח כל אחד מאלו הה' דברים יהיה ד"ה |
|
עתה יהיה לך שאלו השני שטחים יחזיקו ב' צלעות ממרובע אחד שוה הצלעות נצב הזויות ובהיות צלעותיו ה' יהיה שטחו אשר עליו ו"ז כ"ה |
|
ויש לנו שטח הצינסו ושטח הדברים היותם שוים אל ל"ט מפני כי אמרנו צינסו אחד וי' דברים הם שוים אל ל"ט |
|
ויהיה לנו השטח שהוא כ"ה אשר עליו ו"ז מחובר עם ל"ט יעלו לסך ס"ד |
|
ואלו הד' שטחים יהיו מחזיקים בשטח אחד שוה הצלעות ונצב הזויות אשר שטחו הוא ס"ד ועליו ח"ט |
|
וצלעו יהיה שרש ס"ד |
|
וצלע הצינסו שהוא הדבר יהיה ה' פחות |
|
אם כן יהיה לנו שהדבר הוא שרש ס"ד שהוא ח' והוצא מהם ה' שהוא פחות מח' וישאר ג' |
|
א"כ בהיות הדבר ג' הצינסו יהיה ט' רצוני שטחו אשר עליו ב"ג |
|
ושטח כל אחד מן החלקים מהדברים שעליהם ד"ה יהיו ט"ו |
|
א"כ היטב יהיה צינסו אחד ועשרה דברים מראה להיות שוים אל ל"ט מכל אחד משני האופנים האמורים מראים בצורה |
|
|
בפרק השני המורכב הונח צינסו אחד וכ"א יותר שוה לי' שרשים או אמור לי' דברים |
| וזה הפרק אנחנו מראים מהותו מזאת הצורה | |
|
|
|
|
אשר אנחנו מתחילי' ראשונה שיהיה הצינסו המרובע שוה הצלעות ונצב הזויות אשר על שטחו רשמנו א"ב |
|
וכל אחד מצלעיו יהיה שוה לג"ד |
|
ונוסיף לצלע ב"ו שטח אחד מכ"א אשר עליו ב"ז |
|
א"כ אורך השטח מהמספר והצינסו יהיו עשרה שרשים מהצינסו |
|
מפני כי כל אחד מאלו השטחים יהיה מרחבם כשרש הצינסו שהוא ג"ד ב"ו |
|
ואורך שני אלו השטחים הוא כאורך עשרה שרשים והוא קו ג"ז |
|
וזה האורך תחלק לחצי ותמשיך קו אחד עליו ט"כ ויהיה שוה לקו ט"ז |
|
ויהיה זה הקו אם כן ארכו ה' |
|
מפני כי ג"ז ארכו עשרה |
|
וג"ט הוא שוה לט"ז א"כ כל אחד מאלו הקוים יהיה ה' |
|
וכדומה לזה יהיה ד"ח ח"ק אשר הוא שוה לכ"ל |
|
אם כן ט"כ וכ"ל ול"ז וז"ט הם ד' צלעות שוים וכל אחד יהיה ה' |
|
א"כ שטח זה המרובע שעליו כ"ז יהיה כ"ה |
|
וקו ח"כ שוה לקו ו"ט מפני כי ט"ח הוא שוה לו"ב וט"כ הוא שוה לג"ט |
|
תגיע קו אחד מח"נ שוה לג"ו |
|
ומנ' אל מ' שוה לו"ט |
|
ויהיה לך מנ"ק שוה לט"ו או שוה לב"ח |
|
א"כ יהיה לך מרובע אחד שוה הצלעות נצב הזויות אשר צלעותיו יהיו נ"ק ק"ל ל"מ מ"נ |
|
ושטח נ"כ יהיה שוה לשטח ח"ו |
|
ושטח ב"ז או אמור ו"ק הוא יהיה כ"א שהוא השטח שנוסף על הצינסו |
|
א"כ שטח ט"ק ושטח כ"נ יהיו כ"א מפני כי אנחנו עוזבים שטח ו"ח ולוקחים כ"נ שהוא שוה לו"ח |
|
א"כ ישאר השטח המרובע שהוא ל"נ שוה הצלעות היותו ד' וצלעו יהיה שרש ד' |
|
מפני כי שטח כ"ז הוא כ"ה |
|
ושני שטחי ח"ז וכ"נ יהיו כ"א כאשר הראה |
|
א"כ ז"ל שהוא ה' בגרוע ממנו ק"ל שהוא שרש ד' ישאר ק"ז ה' פחות שרש ד' וק"ז הוא שוה לצלע או אמור אל שרש הצינסו |
|
א"כ יהיה לנו שיהיה צלע הצינסו או אמור שרשו אשר ינקב בשם דבר היותו ה' פחות שרש ד' וכשהושב אל מספר מדובר יהיה ג' |
|
והצינסו יהיה הכאתו בעצמו שהוא ט' |
|
א"כ שטח הי' דברים שהוא ד"ז יהיו שלשים |
| עוד רצוני להראותך התקון הנזכר באופן אחר | |
| בהיות כי הדבר שהוא צלע הצינסו יהיה פעמים רבות יותר ממחצית הדברים שרש הנשאר בהוצאת הדברים המספרים או שטחו משטח מחצית כמות הדברים תכפול בעצמו בזה האופן אשר נצייר | |
|
|
|
|
צלע הצינסו יהיה א"ב |
|
וב"ד וג"ד וג"א כל אחד מהם נצב הזויות ושוים זה לזה |
|
שטח זה הצינסו הוא ג"ב |
|
ונוסיף על זה הצינסו כ"א והוא שטח ב"ל |
|
וזה השטח מרחבו קו ב"ו ומאורך כמו הדבר או אמור כצלע הצינסו אשר הוא שוה לו"ל |
|
ויהיו לנו שני אלו השטחים שהם ג"ב וד"ו שוים לעשרה דברים |
|
אשר אלו הי' דברים יש להם מאורך מא' עד ו' |
|
וזה האורך אנו נחצה לשני חלקים שוים בנקודת ה' |
|
א"כ יש לנו א"ה וכן ה"ו יהיו חמשה |
|
ואורך ה"ו נוציא קו אחד ישר מה' אל ע' ויהיה ה"ע חמשה |
|
ונוציא קו אחר ישר מע' אל ח' נכחי לקו ו"ה |
|
ויהיה לנו שטח אחד שוה הצלעות ונצב הזויות עליו ה"ו ו"ח ח"ע ע"ה |
|
ויהיה כל אחד מאלו הצלעות חמשה |
|
ושטח זה המרובע יהיה כ"ה |
|
א"כ שטח ע"ו יהיה ד' יותר מד"ו |
|
וכדומה לזה יהיה לנו שטח ע"ב היותו יותר ד' מד"ח |
|
א"כ יהיה לנו קו אחד ישר מס"פ שוה לע"כ |
|
ועתה יהיה לנו קו ס"נ ופ"ד שוה לע"ח |
|
מפני כי כל כך יעדיף ל"ו על ח"ו כמו שיעדיף ד"ב אל ד"פ |
|
וככה יעדיף א"ב על א"ה כמו ד"ב על ד"פ או ל"ו אל ו"ח |
|
א"כ שטח ב"ס יהיה שוה לשטח |
|
ע"פ הוא שוה לשטח ד"ח |
|
ושטח ד"ו הוא כ"א |
|
א"כ אלו שני השטחים שהם ע"פ כ"ו יהיו כ"א |
|
ושטח ס"ב הוא ד' וצלעו יהיה שרש ד' |
|
וא"ה הוא חמשה מפני כי ה"ו הוא חמשה שהוא אורך מחצית כמות הדברים |
|
אם כן א"ב הוא חמשה ושרש ד' וככה יהיה שוה הדבר |
|
והצינסו הוא הכאתו בעצמו |
|
וכאשר הושב סכומו במספר מדובר יהיה הדבר ז' מפני כי שרש ד' הוא ב' |
|
והצינסו מז' הוא מ"ט שטחו אשר עליו ג"ב |
| We illustrate the third compound chapter this way: | הפרק השלישי המורכב אנחנו נראהו בצורה בזה האופן |
|
נניח שאנחנו יש לנו צינסו אחד שוה לג' דברים או ג' שרשיו וד' דרמי יותר |
|
|
|
|
אנו נניח שהצינסו יהיה שטח על צלעיו א"ב ב"ד ד"ז ז"א ושטחו יהיה א"ד |
|
ונעשה שמז' עד ה' יהיו ג' שרשיו אשר עם ד' דרמי יהיה שוה לכל הסינסו |
|
ונוציא קו אחד נכחי לא"ה |
|
א"כ שטח ה"ד יהיה הג' דברים |
|
ושטח ה"ב יהיה ד' דרמי מפני כי כשנוסיף ד' על ג' דברים הוא שוה לכל הצינסו |
|
אח"כ תחלק קו ה"ז לשני חלקים שוים אשר זה הקו ארכו שלשה דברים ויש לנו מז' עד ח' או מה' עד ח' אחד וחצי |
|
אח"כ תוציא קו אחד מה' אל כ' ואחד מח' אל ט' נכחיים אשר יהיה אורך כל אחד שוה לה"ח שהוא אחד וחצי |
|
א"כ כשנוציא קו ח"ט יש לנו מרובע שוה הצלעות |
|
מפני כי קו כ"ה שוה לה"ח וה"ח שוה לח"ט או לה"כ וכל אחד מאלו הצלעות יהיה אחד וחצי |
|
א"כ שטחו יהיה ב' ורביע והוא שטח ט"ה |
|
עוד רציתי להוסיף על קו ח"ט קו אחד עד נקודת ל' שיהיה שוה לא"ה ויהיה ח"ל שוה לח"א |
|
ועתה תוציא קו אחד מל' בנקודת מ' נכחי לקו ח"א |
|
ויהיה שוה לקו ח"א והוא שוה לח"ל וכן לא"מ |
|
ויהיה לנו מרובע שוה הצלעות והוא שטח ח"מ |
|
ומפני שא"מ הוא שוה לא"ח יהיה מ"ב שוה לח"ז |
|
וח"ז הוא שוה לה"ח וה"ח שוה לח"ט אם כן מ"ב הוא שוה לכ"ט |
|
והיה לנו כי מ"נ הוא שוה לא"ה וא"ה הוא שוה לט"ל א"כ מ"נ הוא שוה לט"ל |
|
ושטח כ"ל יהיה שוה לשטח נ"ב |
|
א"כ שטח א"נ ושטח כ"ל יהיו ארבעה כי שני אלו השטחים א"נ וכ"ל יהיו שוים לשטח ה"ב שהוא ארבעה |
|
אם כן שטח ח"מ יהיה ששה ורביע וצלעו יהיה שרשו מפני כי הוא שטח אחד שוה הצלעות |
|
אם כן צלעו א"ח יהיה שרש ששה ורביע שהוא ב' ורביע |
|
ומח"ז הוא אחד וחצי |
|
שיהיה אם כן כל הקו מא"ז שהוא צלע הצינסו או הדבר יהיה ארבעה במספר או שרש ו' ורביע ויותר א' וחצי כמו שהראית בצורה הרשומה למעלה |
| בכאן נראה האופן והדרך להשיב השאלות אל ההשואות כאשר תוכל לראות | |
Operations with Algebraic Expressions |
|
| Before giving any question, the way and the method are stated, which should be held in order to find the explanation how the questions are restored to the said chapters as well as other chapters that will be given from here on. | קודם שאניח שום שאלה רצוני להגיד הדרך והאופן הצריך להחזיק בו כדי למצוא באור השאלות כיצד יושבו להשואת הפרקים האמורים וג"כ לפרקי' אחרי' אשר יונחו מכאן ולהבא |
| Knowing that what is unknown of the given question should always be defined as a thing or a çenso. | ביודעך לעולם כי אשר אינך יודע מן השאלה המונחת הנך צריך להניח שיהיה דבר או צינסו |
| In any case, it should first be defined as a thing. | ומכל מקום תניח ראשונה שיהיה דבר |
| Yet, many times it would be easier in the question that the unknown term will be çenso. | ופעמים רבות בשאלה יגיעך נקל יותר שהחלק הנעלם יהיה צינסו |
| Since, defining the thing instead of a çenso changes the chapter or the equation. | בהיות כי מהנחת הדבר במקום צינסו יגיע חילוף הפרק או ההשואה |
| As seen above: thing = the side of the çenso, or √çenso | וכמו שהראינו לפנים הדבר הוא צלע הצינסו או שרש הצינסו |
Therefore:
|
ולכן בהכותנו או בכפלנו הדבר בעצמו עושה הצינסו |
|
והדבר כשיוכה בצינסו עושה מעוקב |
| According to these three names, the number is understood in three ways: | ובאלו הג' שמות יובן המספר בג' אופנים |
|
או כפי האורך הקו |
|
או כפי רחב השטח |
|
או כפי העובי שהוא כמות גשמי |
| בהיות כי הראינו לך הדבר והצינסו בצורה שעבר | |
|
רצוני שהדבר הוא אורך צלע הצינסו |
|
והצינסו הוא שטח שוה הצלעות נצב הזויות |
|
רצוני לאמר לך מן המעוקב טבעו כי הוא רחב ואורך וגובה שוים יחד |
|
כמו צורת הקוביא שהוא שוה בגובה וברחב ובארך |
|
וצדדיו יהיו משטחים שוים |
|
וצלעותיו מאורך שוה |
|
וכמו כן זויותיו יהיה שוים זה אל זה |
|
א"כ כל צלע מהמעוקב הוא הדבר |
|
וכל אחד מצדדיו יהיה הצינסו שלו |
|
וראשיותיו הוא המעוקב |
|
א"כ בהכפל דבר בדבר עושה צינסו |
|
ובהכות דבר בצינסו עושה מעוקב |
|
ודבר במעוקב עושה צינסו מצינסו |
| The çenso of çenso can be understood from two aspects: | וזה הצינסו מצינסו אפשר להבינו בשני פנים |
|
בעבור כי צינסו מוכה בצינסו עושה צינסו מצינסו |
|
ודבר מוכה בצינסו עושה צינסו מצינסו |
|
וכשיוכו מספרים בכמות מה מדברים עושה כל כך דברים כמו שעולה כמות הדברי' מוכה במספר |
|
ובהכות כמות מה מצינסי במספר עושה כל כך צינסו כמו שעולה ההכאה ההיא במספר המוכה |
|
ובהכותנו כמות מה ממעוקבים במספר עושה כל כך מעוקבים כמו שעולה הכמות האמור במספר |
|
|
ובהכפל כמות מה מצינסי דצינסי במספר עושה כל כך צינסי מצינסי כמו שעולה כמות הצינסי מצינסי כפולים במספר |
| ועם אלו הד' שמות אשר בארנו שהם דבר וצינסו ומעוקב וצינסו מצינסו יתפשטו הפרקי' אשר יכתבו כפי מה שתראה מכאן ולהבא | |
| ודע כי אם יזדמן לך לכפול מספר ודבר במספר ודבר או מספר וצינסו במספר וצינסו או מספר ודבר במספר פחות דבר או מספר וצינסו במספר פחות צינסו או בכל אופן אחר שיזדמן כפי שנויי ההכפלות שאפשר שיבאו תהיה ממשיך ההכפלה באופן אשר הראית למעלה בכפילת השרשים בהביטך לעולם להמשיך הטבע או העצמויות העושות הכמויות הנקובי' בשם בכפול האחד באחר כמו שכבר הראית קודם וכמו שנראך עתה בכמה המשלים | |
| נניח שרצית לכפול דבר במספר
או דראמא בהיות כי האחדות אשר במספר יקראוהו דראמ' | |
|
|
לכן בכפול ג' דברים בד' דראמי יעלה י"ב דברי' |
|
|
וד' צינסו בג' דראמ' יעלה י"ב צינסו |
|
|
וב' מעוקבי' בה' דראמ' יעלה י' מעוקבי' |
|
|
וה' צינסי מצינסי בג' דראמ' יעלה ט"ו צינסו מצינסו |
| Multiplication of a Number and a Thing by a Number and a Thing | ואם רצית לכפול מספר ודבר במספר ודבר |
|
|
נניח שרצית לכפול ג' וב' דברי' בג' וב' דברים |
| הנך צריך לרדוף הכלל מהכפל בשרשים כפי מה שהראית למעלה | |
| הנך צריך לכפול המספרים זה על זה | |
|
|
שהוא ג' בג' ועולה ט' ושמור |
| אח"כ תכה המספרי' בשתי וערב עם הדברים | |
|
|
שהוא ג' בב' דברים ועולה ו' דברים |
|
|
ואח"כ הג' האחר בב' דברים האחרים ועולה ו' דברים |
|
|
וחברם יחד ויהיה לך י"ב דברים ושמור |
| אח"כ תכפול הדברים זה בזה | |
|
|
דהיינו ב' דברי' בב' דברים ועולה ד' צינסו |
|
|
וחבר הכל יחד ויהיה לך סכומם ט' דראמ' וי"ב דברים וד' צינסי |
| ובזה האופן תרדוף בשאר ההכפלות | |
| בכאן יראה האופן מהחלוק והסקיזארי מאלו המספרי' שהם דבר וצינסו ומעוקב וצינסו מצינסו כאשר תראה תחת זה | |
| עתה רצוני להראות לך באי זה אופן יהיה החלוק והבצוע מאלו השמות שהם דבר וצינסו ומעוקב וצינסו מצינסו | |
|
בהיות כי לחלוק או לבצע הדבר במספר או בדראמ' יגיע דבר |
|
מפני כי בכפול מספר על דבר עושה דבר |
|
ובחלק צינסו על מספר יגיע צינסו |
|
מפני כי הצינסו מוכה במספר עושה צינסו |
|
ובחלק מעוקב על מספר או על דראמ' יהיה המגיע מעוקב |
|
מפני כי המעוקב כשהוכה במספר עושה מעוקב |
|
ובחלוק או לבצע צינסו מצינסו על מספר או דראמ' יגיע צינסו מצינסו |
|
מפני כי בכפול צינסו דצינסו על מספר עושה צינסו מצינסו |
|
ובחלוק דבר על דבר יגיע מספר או דראמ' |
|
ובחלוק צינסו דצינסו יגיע דראמ' |
|
ומעוקב על מעוקב יגיע מספר או דראמ' |
|
ובחלוק צינסו וצינסו דצינסו על צינסו דצינסו יגיע מספר או יהיה דראמ' |
| מפני כי בכפול כל אחד מאלו הכמויות על דראמ' או מספר עושה כמו כן אותו הכמות | |
|
|
וכן לחלוק או לבצע צינסו בדבר יגיע דבר |
|
|
מפני כי הכאת דבר בדבר עושה צינסו |
|
|
ולחלוק מעוקב על דבר יגיע צינסו |
|
|
ולחלוק מעוקב על צינסו יגיע ממנו דבר |
|
|
מפני כי דבר כשהוכה בצינסו או צינסו מוכה בדבר עושה מעוקב |
|
|
ולחלוק צינסו מצינסו על דבר יהיה המגיע מעוקב |
|
|
ולחלוק צינסו מצינסו על צינסו יגיע ממנו צינסו |
|
|
ולחלוק צינסו דצינסו על מעוקב יגיע ממנו דבר |
|
|
מפני כי בכפול דבר במעוקב או צינסו בצינסו או מעוקב בדבר עושה צינסו מצינסו |
| אם כן כל כמות כשהוכפל באחר הנה העולה ממנו כאשר נחלק על אחד מכמויות ההם עושה הכמות האחר | |
| והנה לך למשל במספר | |
|
|
כשהוכפל ד' אחדים על ג' אחדים עושה י"ב אחדים |
|
|
ולחלוק י"ב על הראשון שהוא ד' יגיע ממנו הכמות השני שהוא ג' |
|
|
ובחלוק י"ב בכמות השני שהוא ג' יגיע ממנו הכמות הראשון שהוא ד' |
| דע כי בכאן בקרוב יגלה ההרגל מהו' פרקי' מאלגֵ'בְלֵי אמוגאבאלא כמו שתוכל לראות במשל |
Chapter One |
פרק ראשון |
| When things are equal to numbers.
|
כאשר הדברים יהיו שוים אל המספרי' |
| The number should be divided by the things and the result is the thing.
|
צריך לחלק המספר על הדברי' והעולה יהיה מספר וככה שוה הדבר |
|
שאלה למשל עשה לי מעשרה שני חלקים באופן כי כשהוכה כל חלק בעצמו ויגרע קטון ההכאות מהגדולה ישאר חמשים א"כ כמה יהיה כל חלק מאלו החלקים |
|
זהו כללו כפי אלג'בלי אמוג'בלה |
|
נניח כי אחד מחלקיו יהיה דבר אחד והחלק האחר א"כ ישאר עשרה פחות דבר אחד |
|
עתה תכה דבר אחד בדבר אחד ועולה צינסו אחד |
|
אח"כ תכה החלק האחר שהוא עשרה פחות דבר אחד על עצמו שעולה ק' מספרים או דראמי וצינסו אחד פחות כ' דברים |
|
עתה תוציא החלק האחד המוכה שהוא א' צינסו חוצה מזה הסך מהכאת החלק הגדול בעצמו שהוא ק' מספרים וא' צינסו פחות כ' דברים וישאר ק' מספרים פחות כ' דברים וזה הנשאר הוא שוה לנ' מספרים כפי השאלה הנזכרת |
|
עתה התבונן כי בהיות החלקי' שוים מאחד מהחלקי' יהיו כ' דברים פחות |
|
אם כן בהנתן כ' דברים לכל אחד מהחלקי' כאשר רוצה הכלל מהו' פרקי' האמורים האומ' כאשר יחסר מחלק מה אי זה דבר צריך להוסיף עליו לעולם מה שיחסר וכן צריך להוסיף מחלק האחד כמו לאחר א"כ בהוסיפנו כ' דברים לכל אחד מהחלקים ההם יהיו שוים |
|
ולכן תוסיף כ' דברים על ק' מספרים פחות כ' דברים שיהיה אחד מהחלקים ויהיה ק' מספרים בדיוק |
|
ועל החלק האחר שהוא נ' תוסיף כ' דברים |
|
ועתה יהיה לנו כי ק' מספרים יהיו שוי' לנ' מספרי' וכ' דברי' |
|
|
ועתה צריך להוציא המספר הקטן מהגדול שהוא נ' מק' |
|
|
וישאר מהחלק האחד נ' מספרי' ומן החלק האחר כ' דברי' לבד |
|
|
מפני כי בהיות החלקי' שוים הוצאת נ' מן החלק האחד כמו מהאחר |
|
|
וישאר א"כ נ' מספרי' שוים לכ' הדברי' |
|
|
והם אנו צריכי' לחלק כפי הכלל האמור למעלה המספרי' על הדברים דהיינו נ' על כ' ויבא ב' וחצי וככה שוה הדבר שהוא אחד מחלקי עשרה |
|
|
והאחר ישאר עשרה פחות זה הדבר שהוא ב' וחצי וישאר ז' וחצי וכן הוא החלק האחר |
|
|
א"כ יהיה לך כי אחד מחלקי עשרה הוא ב' וחצי |
|
|
והחלק האחר יהיה ז' וחצי |
| Another example: | עוד רצו' להניח חשבון אחר אל הפרק הזה הראשון |
|
ואומ' כך כי רציתי לקנות ו' אמות בגד מב' מינים אחד מהחלקי' או מיני' מנ"ו דינרים האמה והאחר מס"ז דינרי' האמה |
|
תניח מהכלל האמור ואמור כן אני רוצה דבר אחד מאמות מנ"ו דינרי' |
|
שיהיה נ"ו דברים מדינרי' |
|
ואשאר לקחת ו' אמות פחות דבר אחד מס"ז דינרי' האמה |
|
וכל זה הבגד עלה לי ש"ע דינרי' |
|
|
אבל מפני כי אני מניח שדבר אחד מאמות מנ"ו דינרי' שיהיה נ"ו דברי' מדינרי' |
|
|
ולקחת ו' אמות פחות דבר אחד מס"ז דינרי' שעולה ת"ב דינרי' פחות ס"ז דינרי' |
|
|
אשר הם שוים אל ש"ע |
| ועתה תשוב אל הכלל האמור למעלה שהוא להוציא המספר הקטון מהגדול ולהוסיף על הגורע | |
|
|
ולכן אנו נוציא המספר הקטן מהגדול שהוא ש"ע מת"ב וישאר ל"ב |
|
|
ועתה תגרע הדברי' הכמות הקטון מהגדול שהוא נ"ו מס"ז וישארו י"א דברים שהם יהיה המחלק |
|
|
רצוני לחלק מספר ל"ב על י"א דברים שעולה ב' וי' חלקים מי"א וכן הוא אחד מהכמויו' מהבגד |
|
|
והחלק האחר אם כן הוא השארית עד ו' שהוא ג' וא' מחלק י"א |
| ואשר הוא ב' אמות וי' חלקים מי"א הוא מנ"ו וי' האמה | |
| ואשר הוא ג' אמות וחלק אחד מי"א הוא מס"ז וי' האמה | |
| שעולה סכומם ש"ע דינרים שעושה היטב החשבון שהושם למעלה בעבור הפרק האמור | |
| ואזכירך כי זה החשבון ממש תוכל לעשותו מן שתי ההנחות כמו שתמצא בזה הספר אם תחפש היטב | |
| והנה החשבון האמור נשלם בעבור הפרק הראשון מאלגיבלי | |
|
עוד בעבור הפרק הראשון שאלה תמצא לי מספר אחד אשר אם תחלק שלישיתו בשמינית אחד ממספר יעלה ה' |
|
תניח שהמספר יהיה דבר אחד |
|
|
תקח שלישיתו שהוא שלישית דבר וחלקהו בשמינית מספר |
| ידוע הוא כי כאשר נחלק כמות מה בחלוק מה ומספר החלוק העולה יכפל אחר זה במחלק יגיע מזה אותו הכמות שנחלק ראשונה | |
| וכן אם תחלק שליש אחד מדבר בשמינית אחד ממספר ראוי שיגיע ה' תכה א"כ ה' בשמינית אחד וראוי שיגיע שלישית דבר ואם תכפול ה' בשמינית אחד יעשה ה' שמיניות שיהיו שוים לשלישית אחד מדבר ובזה יהיה לך דבר שוה למספר שהוא שלישית דבר שוה לה' שמיניות ממספר שהוא זאת ההשואה מהפרק הראשון א"כ תחלק המספר על הדבר וזהו ה' שמיניות בשלישית אחד מדבר ובזה יהיה לך דבר שוה למספר ויגיע לך ט"ו שמיניות שהם א' וז' שמניות | |
| ועתה נסה נא תקח השלישית מא' וז' שמיניות ויהיו ה' שמיניות ותחלק בשמינית אחד ויעלה לך היטב ה' ונעשה בעבור הפרק הראשון | |
|
עוד בעבור הפרק הראשון שאלה ג' פוזי וא' זוג סנדלים שוים ל"ב דינרים וו' פוזי וג' זוגות סנדלים שוים פ' דינרים |
|
תניח שהפוזו שוה דבר אחד |
| א"כ ג' פוזי שוים ג' דברי' וזוג סנדלי' שוים ל"ב דינרי' פחות ג' דברי' ואח"כ ו' פוסו שוים ו' דברים וג' זוגו' סנדלים שוים צ"ו דינרי' פחות ט' דברים כאשר הנחת למעלה וזה יהיה שוה לפ' דינרי' תשוה החלקי' תוציא פ' מצ"ו וישאר י"ו עתה הנך יודע כי בחבר יחד ו' דברי' וצ"ו דינרי' פחות ט' דברי' עושים צ"ו פחות ג' דברים שהם שוי' אל פ' א"כ תחלקם כאמור למעלה ותוציא פ' מצ"ו ישאר י"ו אח"כ תתן לכל חלק ג' דברים מפני כי צריך תמיד לשלם במקום שיחסר כאשר הראית למעלה וכמו שאתה רואה בזה החשבון ובזה יהיה לך ג' דברים שוים לי"ו מספרי' תחלק א"כ המספרים על הדברים ויהיה לך ה' ושליש וככה שוה הדבר ואתה הנחת שהפוזו היה שוה דבר אחד א"כ יהיה שוויו ה' דברי' דינרי' ושליש והסנדלי' צריך שישוו בהכרח י"ו דינרי' | |
| והנה נעשה מן הפרק הנזכ' עוד בעבור הפרק האמור | |
|
עשה לי מעשרה שני חלקי' אשר כשנחלק האחד באחר יעשה חמשה |
|
תניח שאחד מהחלקים יהיה דבר אחד |
|
והאחר צריך שיהיה עשרה פחות דבר אחד |
|
|
עתה תחלק עשרה פחות דבר אחד על דבר אחד ויגיע ה' |
|
|
א"כ אם תכפול ה' בדבר אחד יעשה ה' דברי' וזהו שוה אל עשרה פחות דבר אחד |
|
|
להשחית החוב רצו' לשלם במקום החסר נתן לכל חלק החסרון שהוא דבר אחד |
|
|
ויהיה לנו עשרה מספרי' ויהיה לנו עשרה בלי חשבון והאחר שהוא ו' דברים יהיה ו' דברי' והנה כי יש לנו שעשרה שוה לו' דברים |
| א"כ נרדוף הכלל ונחלק עשרה על ו' ויהיה העולה א' וב' שלישיו' וככה שוה הדבר ואנחנו הנחנו שהראשון היה דבר אחד א"כ היה א' וב' שלישיו' | |
| והחלק האחר היה הנשאר עד עשרה שהוא ח' ושליש | |
| ואם רצית לנסותו תחלק ח' ושליש על א' וב' שלישי' והיטב יצא ה' ונעשה בעבור הפרק הראשון |
Chapter Two |
פרק שני |
| Its nature is as written right below | וטבעו כמו שכתו' תחת זה מיד |
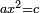
|
כאשר הצינסי יהיו שוים אל המספרים |

|
צריך לחלק המספרים על הצינסי והעולה יהיה מספר וככה שוה הצינסי |
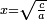
|
ושרש העולה מזה כן ישוה הדבר |
|
שאלה עשה לי זה החשבון הנה ב' אנשי' אשר להם עשרה דראמ' מעות ואיני אומ' לך כמה דראמ' יש לכל אחד לבדו אבל כשהוכפלו הדרמ' אשר לאחד ולאחר בעצמם יעשו כ' ורביע |
| Following the rule: | תעשה כמו שאומ' הכלל |
|
תניח כפי אלגיברא שאחד מהחלקים יהיה דבר אחד וה' מספרים |
|
והחלק האחר ישאר ה' פחות דבר אחד |
|
עתה אקח ההבדל שיש בין החלק האחד לאחר רצו' מדבר אחד וה' מספרים אל דבר אחד פחות ה' מספרים פחות דבר אחד וזה ההבדל יהיה ב' דברי' |
|
וסבת זה |
|
כי אחד מהחלקי' הוא דבר אחד וה' מספרים |
|
והחלק האחר הוא ה' פחות דבר אחד |
|
א"כ אחד מהחלקי' הוא ב' חלקי' יותר מהאחר |
|
|
ואם תרצה אראך זה באופן אחר בהוציאך ה' פחות דבר אחד מה' ודבר אחד ואם וישאר ב' דברי' וככה הוא הדרמ' האמור דהיינו כמה הוא החלק האחד גדול מהאחר |
|
|
וזאת הדרמ' רצוני הב' דברי' תכפלהו בעצמו יעלה ד' צינסי |
|
|
ואלו הד' צינסי הם שוים אל כ' ורביע |
|
|
עתה תחלק המספרי' על הצינסי שהם כ' ורביע על ד' ויעלה ה' וחלק אחד מי"ו וככה שוה הצינסי |
|
|
ושרש ה' וחלק מי"ו הוא הדבר וזה השרש הוא ב' ורביע |
|
ואתה הנחת שאחד מהחלקי' היה דבר אחד וה' מספרי' א"כ יגיע להיות ב' ורביע וה' שעולה ז' ורביע וככה הוא אחד מהחלקי' |
|
והחלק האחר הונח ה' פחות דבר אחד שהוא ה' פחות ב' ורביע וישאר ב' וג' רביעי' וככה הוא החלק האחד מעשרה |
|
|
א"כ יהיה לך אחד מהחלקים ז' ורביע והאחר ב' וג' רביעי' |
|
ונעשה עם הפר' הב' |
| Although this calculation was defined by the first method of the algebra, it was not defined by the general conventional method, as the conventional method defines the unknown as one thing, while here it was defined as a thing plus five numbers. | הנני מודיעך כי אע"פ שזה החשבון הונח באופן הראשון מהאלזיבר' לא הונח באופן הכללי הנהוג בהיות כי הכללי הנהוג מניח אותו שאינו ידוע דבר אחד ואנחנו הנחנו דבר אחד וה' מספרי' בעשות מעשרה אותם שני חלקים שנשאלו למעלה |
| The reason for that is: | וסבת זה למה היא זו |
|
כי בהניח אחד מהחלקים מעשרה שהיה דבר אחד |
|
והחלק האחר היה עשרה פחות דבר אחד |
|
והדרמ' היתה אז פחות ב' דברים מפני כי להוציא דבר אחד מעשרה פחות דבר אחד ישאר עשרה פחות ב' דברים וככה הוא הדרמ' רצוני אשר הוא החלק האחד הגדול מחבירו |
|
וברצות לרדוף בחשבון כפי התנאים האמורי' להשיבו אל התכלית יבא אל הפרק הה' בהכרח |
|
ואנחנו רצינו להשיבו בפרק השני ולכן שמנו אל דבר אחד וה' מספרים כאשר הראה למעלה |
|
עוד תדע כי אם רצית להוציא החלק האחר שהוא עשרה פחות דבר אחד חוצה מהחלק האחר האמור דבר אחד יהיה הנשאר ב' דברים פחות עשרה והיית יכול לאמר שזה יהיה ההבדל באמת |
|
ולרדוף עוד בזה האופן הרושם החשבון יצא גם כן מהפרק החמישי |
The things [ ] would have been derived differently, but the parts [ ] would have been derived differently, but the parts [ ; ;  ] would have been well defined, as will be shown below in chapter five. ] would have been well defined, as will be shown below in chapter five.
|
אבל הדברים היו באים שונים זה מזה והחלקי' היו באים בזה עשויים היטב כפי מה שנראך בדרכינו לפנים בפרק הה' |
| בהיות כי מהפרק האמור יענה פעמים רבות חשבונות מה מספרים ושרשים וחשבונו' מה מספרי' פחות שרשים ואחרי' יוכלו לענות יותר שרשי' ואחדים פחות שרשים ונעשה מהפרק השני | |
| Another one of chapter two: | עוד מהפרק השני |
|
תמצא לי מספר אחד כי כשהוכה בעצמו ואח"כ מחצית אותו מספר יוכה בעצמו ואלו שתי ההכפלו' תחבר יחד יעשה עשרה |
|
תניח כי המספר יהיה דבר אחד |
|
|
תכפלהו בעצמו ועושה צינסו אחד |
|
|
אח"כ תכפול מחצית הדבר ויעשה רביע אחד מצינסו |
|
|
תחברם יחד ויעשה צינסו אחד ורביע אחד מצינסו והוא שוה לעשרה |
|
|
עתה תחלק המספר על הצינסו דהיינו עשרה על א' צינסו ורביע ויעלה שרש ח' |
|
|
ושורש ח' ישוה הדבר ואתה הנחת שהמספר היה דבר אחד א"כ היה שרש ח' |
| Solved according to chapter two. | ונעשה מהפרק השני |
| Another one of chapter two: | עוד מהפרק השני |
|
תמצא לי מספר אחד שכאשר הוכה על ג' רביעיו יעשה מ' |
| Following the rule: | תעשה כמו שאומ' הכלל |
|
תניח שהמספר יהיה דבר אחד |
|
|
תקח ג' רביעיו שהם ג' רביעים מדבר תכפלם בדבר אחד ועושה ג' רביעים מצינסו שהם שוים אל מ' מספרי' |
|
|
תחלק המספרי' על הצינסי דהיינו מ' על ג' רביעי' ויגיע לך נ"ג ושליש |
|
|
וכן שורש נ"ג ושליש שוה הדבר ואתה הנחת שהמספר היה דבר אחד א"כ היה שרש נ"ג ושליש |
| This number is inexpressible | וזה המספר הוא בלתי מדבר ואי אפש' לבטאות בו |
| Solved according to chapter two. | ונעשה מהפרק השני |
| Another one of chapter two: | עוד מהפרק השני |
|
תמצא לי מספר אחד אשר כשהוצא ממנו שלישיתו ורביעיתו והנשאר יוכה בעצמו יעשה י"ב |
|
תניח שהמספר היה דבר אחד |
|
|
והשליש והרביע מדבר הוא ז' חלקים מי"ב |
|
|
הוציאם מדבר אחד ישאר ה' חלקים מי"ב מדבר |
|
|
עתה תכפול ה' חלקים מי"ב בדבר על ה' חלקי' מי"ב בדבר ועושה כ"ה חלקים מקמ"ד בצינסו שהם שוים אל י"ב |
|
|
א"כ תחלק י"ב על כ"ה חלקים מקמ"ד בצינסו ויגיע לך ס"ט וג' חלקים מכ"ה |
|
|
ושרשו ישוה הדבר ואתה הנחת שהמספר היה דבר אחד א"כ היה שרש מס"ט וג' חלקים מכ"ה |
| Solved according to chapter two. | ונעשה מהפרק השני |
Chapter Three |
הפרק השלישי |
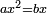
|
וטבעו כאשר הצינס' יהיו שוים לדברים |
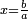
|
צריך לחלק הדברים בצינסי והמגיע הוא מספר וכך שוה הדבר |
|
תמצא לי מספר אחד אשר כשהוכה בשני שלישיו יעשה ג' פעמי' כמו המספר הנמצא |
| Following its rule: | תעשה כמו שאומ' הכלל שלו |
|
תניח שהמספר יהיה דבר אחד |
|
|
ועתה תקח שני שלישיו שהוא ב' שלישי דבר |
|
|
ותכה ב' שלישי דבר בדבר ועולה ב' שלישי צינסו |
|
|
ואלו ב' שלישי צינסו הם שוים לשלשה פעמים המספר הנמצא דהיינו ג' דברים שוים לב' שלישי צינסו |
|
אשר אנו צריכי' לחלק כפי הכלל האמור למעלה שהוא לחלק הדברים על הצינסי |
|
|
שהוא ג' בב' שלישיו שיגיע ממנו ד' וחצי וככה שוה הדבר רצוני המספר הנשאל |
| Another one of the said chapter: | עוד מהפרק האמור |
|
תמצא לי ב' מספרים שיהיה האחד חלק מהאחר כמו שהוא ב' מג' ויעשה כשהוכו האחד באחר כמו כאשר חוברו יחד |
|
תניח שהראשון היה ב' דברים |
|
והשני ג' דברים |
|
|
עתה תחבר ב' דברי' עם ג' דברים ועושים ה' דברים |
|
|
עתה תכה ב' דברי' בג' דברי' ועושים ו' צינסי |
|
|
עתה יש לנו שה' דברים הם שוים לו' צינסו |
|
תשוב אל הכלל האמור למעלה שהוא לחלק הדברי' על הצינסי |
|
|
דהיינו ה' על ו' שעולה ה' ששיות וככה שוה הדבר |
|
ואתה הנחת שהמספר הראשון היה ב' דברי' א"כ היה א' וב' שלישי' |
|
ועוד הנחת שהשני היה ג' דברי' א"כ היה ג' פעמי' ה' ששיות שעולים ב' וחצי וככה היה המספר השני |
| Solved according to chapter three. | ונעשה מהפרק השלישי |
| Another one of chapter three: | עוד מהפרק השלישי |
|
תמצא לי מספר אחד שמחציתו מוכה בעצמו יעשה כמו אם הוכה המספר בעשרים |
|
תניח שהיה המספר דבר אחד |
|
|
תקח מחציתו שהוא חצי דבר |
|
|
תכהו בעצמו ועושה רביע צינסו |
|
|
אח"כ תכפול דבר אחד בעשרים ועושה כ' דברים שהם שוים לרביע אחד מצינסו |
|
ועתה תחלק הדברים על הצינסי |
|
|
ויעלה פ' וככה שוה הדבר ואתה הנחת שהמספר היה דבר אחד א"כ היה הוא פ' |
| Solved according to chapter three. | ונעשה מהפרק השלישי |
| Another one of chapter three: | עוד מהפרק השלישי |
|
תמצא לי מספר אחד שיעשה הכאתו בעצמו כל כך שעושה אם נחלק על ק' |
|
תניח שהמספר היה דבר אחד |
|
|
תכהו בעצמו ועושה צינסו אחד |
|
|
אח"כ תחלק דבר אחד בק' ויעלה חלק אחד מק' בדבר שהוא שוה לא' צינסו |
|
|
תחלק חלק אחד מק' על אחד ויעלה עוד חלק אחד מק' וכך שוה הדבר ואתה הנחת שהיה המספר דבר אחד א"כ היה חלק אחד מק' |
| Solved according to chapter three. | ונעשה מפרק ג' |
Chapter Four |
הפרק הרביעי |
| והוא המורכב ראשון טבעו | |
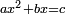
|
כאשר הצינסי והדברי' יהיו שוים אל המספר |
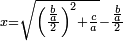
|
צריך לחלק כל ההשואה על הצינסי אח"כ תחלק מחצית הדברים לחצי |
|
עשה לי מעשרה שני חלקי' שכשהוכה החלק הקטן בעצמו יעשה כל כך כמו שיעשה הכאת רביע הגדול בעצמו |
| Following the rule: | תעשה כמו שאומ' הכלל |
|
תניח שאחד מהחלקי' יהיה דבר אחד |
|
והאחר צריך שישאר עשרה פחות דבר אחד |
| אח"כ תוכל להניח אי זה מהם שתרצה בעד הגדול | |
| ואנחנו נניח כי החלק הקטן יהיה דבר אחד | |
| וסבת למה היא זאת אם הנחת שהחלק הגדול היה דבר אחד היה יוצא החשבון אל הפרק החמישי מפני ההשואה | |
| ואנחנו מבקשים להשיבו אל הרביעי אשר אנחנו בו | |
|
|
ולכן אנחנו נכה דבר אחד בעצמו בעד החלק הקטן ועושה א' צינסו |
|
|
עתה נקח הרביע מהגדול שהוא עשרה פחות דבר אחד ויהיה ב' וחצי פחות רביע אחד מדבר |
|
|
וזה כאשר הוכה בעצמו עולה ששה מספרים ורביע וחלק א' מי"ו בצינסו פחות דבר אחד ורביע |
|
|
וזה הכפל הוא שוה לצינס' אחד שהוא כפל החלק הראשון בעצמו |
|
|
ועתה צריך אתה לרדוף בזה האופן בהיות כי כל אחד מהחלקי' יש לו צינסו הנך צריך להוציא הצינסו שבחלק הקטון מהצינסו שהם בגדול דהיינו להוציא מכל אחד מהחלקי' כל כך צינסו כמו שהוא החלק הקטון וישארו החלקים שוים זה לזה והראשון ישאר ט"ו חלקים מי"ו בצינסו |
| עתה הנך צריך לחבר אל כל אחד מהחלקים כל כך דברים כמו שיש לאחד מהחלקים פחות | |
|
|
א"כ תחבר לחלק אשר לו דבר אחד ורביע מפחת אותו עצמו שיש לו מפחת ויהיה לך אין כלום מפחת ואל החלק האחר תחבר אותו בעצמו שהוא דבר אחד ורביע ויהיה לך אחד מהחלקים בהיות מוצאים הצינסו ומחוברי' הדברים כפי האמור למעלה שוה לאחר רצוני לראשון שהוא ט"ו חלקי' מי"ו בצינסו ודבר אחד ורביע שוה לחלק האחר שהוא ו' ורביע והוא השני |
|
|
עתה תחלק כל ההשואה על הצינסו שהוא ט"ו חלקי' מי"ו |
|
|
ויעלה אחד מהחלקים א' צינס' ודבר אחד ושליש שוה לו' מספרי' וב' שלישי' שהם החלק השני |
| ועתה אנחנו צריכי' לחלק כפי הכלל האמור קודם הפרק האמור רצו' הדברי' לחצי שיהיו אחד מהחצאים יהיו ב' שלישי' ואלו הב' שלישים צריכי' אנו לכפול בעצמם אשר יעלו ד' תשיעיות | |
|
| |
|
|
והחלק האחר הוא הנשאר עד עשרה שהוא עשרה וב' שלישי' פחות שרש ז' וא' תשיעי' |
|
|
ומפני שז' ותשיעית יש לו שרש אשר הוא ח' שלישי' והם ב' וב' שלישי' |
|
|
והאמור ראוי להיות ב' שלישי' פחות מהשרש האמור א"כ תוציא ב' שלישי' מב' וב' שלישי' וישאר ב' וככה הוא החלק הראשון |
|
|
ואלו הב' תוציא מעשרה וישארו ח' וככה הוא החלק השני |
| עתה רצוני להזכירך שאם השאלה תאמ' שכך עושה החלק הראשון מוכה בעצמו כמו רביע החלק הגדול ה מוכה בעצמו בחלוף מה שאומ' כמו שיעשה הכאת רביע הגדול בעצמו הנה תהיה שאלה אחרת מפני ההבדל אשר יש בין מאמ' למאמ' כי באמרנו רביע הגדול מוכה בעצמו צריך לכפול כל החלק בעצמו וממנו ילקח הרביע ובאמרנו כמו שהוא הכאת רביע הגדול בעצמו צריך לקחת ראשונה החלק הגדול ואותו הרביע תכהו בעצמו וזהו המובן מחשבוננו | |
|
עוד בעבור הפרק הרביעי תמצא לי מספר אחד שכאשר הוכה בעצמו ואחר יוכה בח' ומקובץ שתי אלו ההכאות יחד יהיה ל"ג |
|
|
תניח שהמספר היה דבר אחד |
|
|
תכהו בעצמו עושה א' צינסו |
|
|
אח"כ תכהו בח' ועושה ח' דברים |
|
|
תחברם יחד ויהיה לך א' צינסו וח' דברים שוים אל ל"ג |
| הנך צריך לחלק על הצינסי דהיינו להשיב אל צינסו אחד על א' צינסו ועולה עוד א' צינסו וכיוצא בזה תחלק ח' על אחד ויגיע עוד ח' ותחלק ל"ג על אחד יגיע עוד ל"ג | |
| עתה תחצה הדברי' שהם ח' ויהיה לך ד' תכהו בעצמו ועושה י"ו | |
|
| |
| וזה נעשה מהפרק הרביעי שהוא המורכב ראשון | |
|
עוד בעבור הפרק הרביעי תמצא לי מספר אחד שכשהוכה שלישיתו בעצמו ותחובר ההכאה ההיא על המספר יעשה י"ב |
|
|
תניח שהמספר יהיה דבר אחד |
|
|
תקח שלישיתו שהוא א' שליש מדבר |
|
|
תכהו בעצמו ועושה א' תשיעית מצינסו |
|
|
תחברהו עם דבר אחד ויהיה לנו א' תשיעית מצינסו ודבר אחד שוה אל י"ב |
|
|
עתה תחלק הצינסי דהיינו כל החלקים על א' תשיעית |
|
|
ויהיה לך א' צינסו וט' דברי' וק"ח דראמי דהיינו מספרי' וזאת ההשואה היא א' צינסו וט' דברים שהם שוים אל ק"ח דראמ' |
| עתה תחצה הדברי' ויהיה לך ד' וחצי תכפלם בעצמם ויעלה כ' ורביע | |
|
| |
| ונעשה מהפרק הרביעי שהוא גם כן המורכב ראשון | |
| עוד בעבור הפרק הרביעי אחד הלוה לאחר כ' ליטר' בעד ב' שנים לעשות ראש משנה לשנה ובסוף שתי שנים השיב לו בין קרן וריוח ל' ליטר' אשאל לאי זה חשבון הולוה הליט' לחדש | |
| תניח שהולוה לא' דבר ממעו' לחדש | |
| וישוו לשנה אחת י"ב דברי' | |
| תקח א' מעשרים מליט' אחת שהיא היטב כ' דינרים שהוא דינר אחד א"כ נאמ' אנחנו עוד חלק מעשרים כאמור למעלה ויהיה לך כ' ליט' ודבר אחד עתה תקח החלק אחד מעשרים ודבר אחד שהוא דבר אחד וחלק אחד מעשרים בצינסו ויהיה לך סכומם שיהיה כ' ליטר' וב' דברים וחלק אחד מכ' בצינסו שהם שוים אל ל' הוצא כ' מל' וישאר עשרה עתה תחלק על הצינסי כל החלקים ויגיע לך א' צינסו ומ' דברים שיהיו שוים למאתים מספרי' | |
| א"כ תחצה הדברים שהם מ' ויהיה לך כ' תכפלם בעצמם ויהיה לך ד' מאות | |
|
| |
| ואתה הנחת שהליטר' הולותה לא' דבר לחדש א"כ הולותה לשרש ו' מאות פחות כ' ממעות לחדש | |
| והוא נעשה מן הראשון המורכב | |
Chapter Five |
הפרק החמישי מן האלזיברא |
| We show you its nature in it and it is the second compound [type of equations] | נראה לך בו טבעו והוא המורכב השני |
| When squares and numbers are equal to things.
|
כאשר הצינסי והמספרים יהיו שוים אל הדברי' |
| The whole equation should be divided by the number of the squares.
|
צריך לחלק כל ההשואה על כמות הצינסי |
| Then, [the number of] the things is divided in half. | ואח"כ לחלק הדברים לחצי |
| One of the halves is multiplied by itself. | ולכפול אחד מן החצאים בעצמו |
| This product is subtracted from the number. | ולהוציא מן הכפל ההוא המספרים |
| Add the root of the remainder to the other half of [the number of] the things and this is equal to the thing. | ושרש הנשאר תחבר אל המחצית האחר מהדברי' וככה שוה הדבר |
| Sometimes, in certain calculations, when the equation is related to this chapter, the thing is half [the number of] the things minus the root of the remainder from the subtraction of the number from the product of the other half [the number of] the things.
|
ופעמים בחשבונות מה בבא אל ההשואה מזה הפרק הדבר יהיה מהמחצית מהדברים פחות שרש הנשאר מהוצאת המספר מכפל המחצית האחר מהדברים |
| Some calculations can be answered both ways, i.e. half [the number of] the things plus a root of the stated remainder, or half [the number of] the things minus a root of the stated remainder
|
וחשבונות מה יוכל לענות בם בשני האופנים דהיינו מחצית הדברים ויותר שרש משארית האמור או מחצית הדברים פחות שרש השארית האמור |
|
והנה המשל עשה לי מעשרה שני חלקים שכאשר הוכה ההבדל שבין זה לזה בעצמו יעשה עשרים ורביע |
| Following the rule: | תעשה כמו שאומר הכלל |
|
אנחנו צריכין להניח שאחד מהחלקים יהיה דבר אחד |
|
והאחר ישאר עשרה פחות דבר אחד |
|
|
עתה תקח ההבדל שבין זה לזה שהוא שני דברים פחות עשרה |
|
ואם רצית לדעת כיצד יהיה ההבדל ב' דברים פחות עשרה תוסיף ב' דברים פחות עשרה על עשרה פחות דבר אחד שהוא החלק השני ויהיה לך דבר אחד |
|
|
א"כ הנך רואה היטב כי להוציא מדבר אחד עשרה פחות דבר אחד ישאר ב' דברים פחות עשרה וכן הוא ההבדל מה שהחלק האחד גדול מהאחר |
|
|
וזה ההבדל שהוא ב' דברי' פחות עשרה תכפול בעצמו ועולה ק' מספרים וד' צינסו פחות מ' דברי' שהם ישוו אל כ' ורביע |
|
עתה צריכי' אנו לחלק כל ההשואה על כמות הצינסי דהיינו על ד' כפי הכלל האמור למעלה |
|
|
שעולה א' צינסו וכ"ה מספרים פחות עשרה דברים שוים לה' מספרים וחלק מי"ו |
|
|
עתה צריכי' אנו להוציא המספרים הקטנים מכל אחד מהחלקים שהם ה' וחלק מי"ו |
|
|
וישאר לאחד מהחלקים לא כלום ולאחר ישאר י"ט וט"ו חלקים מי"ו |
|
|
ועתה תוסיף על כך אחד מהחלקים כל כך דברים כמו שאחד מהחלקים יש לו פחות |
|
|
ואחד מהם יהיה לו לא דבר ולחלק האחר יהיה לו עשרה דברים |
|
|
א"כ יהיה הנשאר לאחד מהחלקי' א' צינסו וי"ט מספרים וט"ו חלקים מי"ו שוה לעשרה דברים דהיינו לחלק האחר |
| ועתה הנני מודיעך כי ההמשך שעשינו כאשר היה לנו ק' מספרי' וא' צינסו פחות מ' דברי' שוים אל כ' מספרים ורביע היה לו זה הפחיתות שההשואה לא הסכימה עם הכלל הנתון למעלה | |
| ואמות זה הוא כי לא הוצאו עדיין המספרי' הקטנים מכל אחד מהחלקי' גם לא נוספו הדברים אע"פ שהחשבון יצא לפעל אמתי אנחנו חלקנו על כמות הצינסי קודם הזמן כמו שנראה בכלל הפרק האמור | |
|
|
וראוי לנו להחזיק בזה האופן שאנחנו צריכי' להוציא המספר הקטן שהוא כ' ורביע מכל אחד מהחלקים |
|
|
וישאר לאחד מהחלקים לא שום מספר ואל האחר ע"ט וג' רביעי' וד' צינסי פחות מ' דברים |
|
|
ואח"כ היה ראוי להוסיף מה שגורע מאחד מהחלקים לכל אחד מהחלקים |
|
|
היה נשאר ולאחד מהחלקים נשאר ד' צינסו וע"ט וג' רביעי' ולחלק האחר כ' דברים |
|
|
שהוא היטב מסכים לכלל הקודם האומ' הצינסי והמספרים שוים אל הדברים |
|
|
כי בחלוק על הצינסי עולה היטב כך כמו שעולה באופן האחר שהוא א' צינסו וי"ט מספרים וט"ו חלקים מי"ו שוים לי' דברים |
| עתה נחצה הדברים בהמשכה אחר הכלל ויהיה לנו ה' ואלו הה' נכפלם בעצמם ועולה כ"ה | |
|
| |
|
|
והחלק האחר הוא הנשאר עד עשרה שהוא ב' וג' רביעים |
| וזה החשבון נענה מן האופן הראשון שהוא מחצית הדברי' ויותר שרש הנשאר האמור | |
| ובאופן אחר אי אפשר לענות בו בהיות שהונח אל ההשואה האמורה למעלה בקחת ההבדל באופן האמור | |
|
|
וסבת זה היא כי אם אמרת היות ההבדל באופן אחר שיוכל להיות רצוני עשרה פחות ב' דברים אפשר שיעלה החשבון כאמור דהיינו בפחות מה שהיה יכול להיות הדבר אם היה יכול להיות החלק הקטן |
| וזה הדבר אי אפשר לעשותו בהיות ההבדל ב' דברים פחות עשרה | |
|
|
בעבור שב' דברים צריכי' להיות יותר מעשרה |
|
|
אם עשרה צריך להוציא מאותו שעולה ב' דברים |
|
|
והנה המשל אם הדבר יבא להיות ה' ושרש ה' וחלק מי"ו כי כאשר הושב אל מספר יבא להיות ז' ורביע כל החלק |
|
|
א"כ ב' דברים יבא להיות י"ד וחצי |
|
|
וההבדל נעשה ב' דברים פחות עשרה א"כ תוציא עשרה מי"ד וחצי וישאר ד' וחצי והנה ההבדל יבא להיות ד' וחצי |
|
|
וזאת ההכאה יעשה היטב כ' ורביע |
|
|
ואם תאמ' שהדבר יהיה ה' פחות שרש ה' וחלק מי"ו אשר כשהושב אל שלם יבא להיות ב' וג' רביעים |
|
יהיה בלתי אפש' כי ב' דברים כאלה עשרה יוכל להוציא |
| ולכן אי אפש' לענות בעשות ההבדל ב' דברים פחות עשרה כי אם מהיותר כפי מה שענינו | |
| עוד בעבור הפרק החמישי | |
|
עשה לי מעשרה שני חלקים שכשהוכה החלק הקטן בעצמו ותוציא העולה מזה מהכאת ההבדל שיש בין החלקים בעצמו ישאר ל"ב אשאל כמה יבא להיות מהחלקים |
| Following the rule: | תעשה כמו שאומ' הכלל |
|
תניח שהחלק הקטון יהיה דבר אחד |
|
והאחר יבא להיות השארית עד עשרה שהוא עשרה פחות דבר אחד |
|
|
עתה תכה החלק הקטן שהוא דבר אחד בעצמו ויעלה א' צינסו |
|
|
עתה תקח ההבדל אשר בין הקטן והגדול שהוא ב' פחות עשרה דברים |
|
|
ותכהו בעצמו ועלה ד' צינסו וק' מספרים פחות מ' דברים |
|
|
עתה תוציא הכאת החלק הקטן שהוא א' צינסו מהכאת ההבדל בעצמו שעשינו שהיא ד' צינסי וק' מספרי' פחות מ' דברים וישאר עשרה צינסו וק' מספרים פחות מ' דברי' שוים אל ל"ב מספרים כפי השאלה הנתונה למעלה |
|
|
עתה צריכים אנו להוציא המספר הקטון מכל אחד מן החלקים שהוא ל"ב |
|
|
וישאר אל אחד מהחלקים אין מספר ולחלק האחר ג' צינסו וס"ח מספרים פחות מ' דברים |
|
|
אח"כ תוסיף מ' דברים שהוא פוחת לאחד מהחלקים |
|
|
ולכל חלק ישאר השואתו דהיינו לחלק האחד ג' צינסי וס"ח מספרים ולאחר מ' דברי' והנה יש לנו כי ג' צינסי וס"ח מספרי' הם שוים למ' דברי' |
|
עתה צריכי' אנו לחלק כל ההשואה בכל כמות הצינסי שהם ג' כפי הכלל האמור למעלה |
|
|
ויגיע לנו א' צינסו וכ"ב מספרים וב' שלישי' שוים לי"ג דברים ושליש |
|
|
עתה שהושבה אל א' צינסו תחלק הדברים לחצי שעולה ו' וב' שלישי' ותכם בעצמם ועולה מ"ד וד' תשיעיות |
| והושבה מן האופן השני שאמר הפרק החמישי | |
|
|
והחלק האחר יבא להיות הנשאר עד עשרה שהוא ג' ושליש ושרש כ"א וז' תשיעיות |
|
|
ועתה הנני מודיעך שאתה אם לקחת ההבדל באופן האחד שאפש' לקחתו כפי מה שהראית קודם באופן הראשון שיבא להיות עשרה פחות ב' דברי' היה בא המענה כדומה לזה האמור למעלה |
|
ובאופן אחר אי אפש' לענותה בהניח שהחלק הקטן הוא דבר אחד בבא החשבון אל זה הראש |
| א"כ החשבון אי אפשר להגיע תשובתו כי אם מספר פחות שרש | |
|
|
מפני שהחלק הקטן מוכה בעצמו צריך שיעשה פחות מההבדל מוכה בעצמו |
|
אחר שבהוצאת הכאת הקטן מן הכאת ההבדל צריך שישאר ל"ב |
| ומפני זה החשבון האמור לא יתכן לשומו בזה הפרק כי אם בשני האופנים האמורים למעלה | |
|
ומפני כי באמרנו היות הדבר מספר ושרש היה נראה שהחלק הקטן שהוא ששה וב' שלישי' ושרש מכ"א וז' תשיעיות יהיה יותר משני החלקים שהם ראויים להיות עשרה |
|
וזה דבר נמנע שהחלק יהיה גדול מהכל |
|
ואם רצית לראות ראית זה מבוארת |
|
|
תקח השרש מכ"א וז' תשיעיות שהוא ד' וב' שלישי' ותוציאם מו' וב' שלישי' ישאר ב' |
|
|
והחלק הגדול יבא להיות הנשאר עד עשרה שהוא ח' |
|
|
וההבדל יהיה ו' כשתפיל ב' מח' |
|
|
תכה ו' בעצמו ועושה ל"ו |
|
|
עתה תכה החלק הקטן שהוא ב' בעצמו ועושה ד' |
|
|
תוציאם מל"ו וישאר ל"ב כפי השאלה האמורה למעלה |
| עוד בעבור הפרק האמור למעלה | |
|
תעשה לי מעשרה שני חלקים שכשהוכה האחד באחר יעשה כ"א |
|
|
זהו כלל נהוג תניח שאחד מהחלקים יהיה דבר אחד |
|
|
והאחר ישאר עשרה פחות דבר אחד |
|
|
תכה דבר אחד בעשרה פחות דבר אחד ועולה עשרה דברים פחות א' צינסו |
|
|
ואלו העשרה דברים פחות א' צינסו הם שוים אל כ"א |
|
|
עתה צריך אתה לתת א' צינסו לכל אחד מהחלקים |
|
|
ויהיה לך עשרה דברים שוים לא' צינסו וכ"א מספרים |
|
עתה תחצה הדברים ויגיע ה' |
|
תכה ה' בעצמו ועושה כ"ה |
|
עתה תוציא המספר שהוא כ"א מכ"ה וישאר ד' |
| ושרש אלו הד' תחבר מהחלק האחר שהוא ממחצית הדברים שהוא ה' ויהיה לך ה' ושרש ד' | |
| או אם תרצה אמור ה' פחות שרש ד' וכן ישוה הדבר | |
|
| |
| והנשאר עד עשרה הוא החלק האחר | |
| א"כ הנך יכול לענות ביותר ובפחות מה ששוה הדבר | |
| עתה ראית כל התשובות אשר אפש' לעשותם בעבור הפרק החמישי האמור | |
| עוד בעבור הפרק החמישי האמור | |
|
עשה לי מעשרה ב' חלקים אשר כשהוכה האחד בג' יעשה כך כמו שעולה הכאת האחר בשרש ח' |
|
|
תניח שאחד מהחלקים יהיה דבר אחד |
|
|
והאחר מחוייב שיהיה עשרה פחות דבר אחד |
|
|
עתה תכה ג' בדבר אחד ועושה ג' דברים |
|
ותכה שרש ח' בעשרה פחות דבר אחד שעושה ק' פחות כ' דברים ויותר א' צינסו |
|
|
עתה תשוה החלקים ותן לכל חלק ק"ס דברים |
|
|
ויהיה לך קס"ג דברים להיות שוה לת"ת מספרי' וח' צינסי |
|
תחלק א"כ כל דבר על הצינסי להשיב כל דבר אל א' צינסי |
|
|
שיגיע מזה א' צינסו וכ' דברים וג' שמיניות שהוא ק' מספרים |
|
עתה תחצה הדברים שהם עשרה וג' חלקים מי"ו |
|
תכפלם בעצמם שעושה ק"ג ור"א חלקים מרנ"ו |
|
תפיל מהם המספר שהוא ק' ישאר ג' ור"א חלקים מרנ"ו |
| ושרש זה הג' ור"א חלקים מרנ"ו ויותר מחצית הדברים שוה הדבר | |
|
| |
| ואתה הנחת שאחד מהחלקי' היה דבר אחד א"כ היה שרש ג' ור"א חלקים מרנ"ו יותר ממחצית הדברים | |
|
והחלק האחר היה הנשאר עד עשרה שהוא שרש ג' ור"א חלקים מרנ"ו פחות מן עשרה וג' חלקים מן י"ו |
| אני האמנתי שזה היה נעשה היטב ועתה בעשות הנסיון מצאתיהו משובש ועתה אעשנו כראוי להיות ואפש' להוליכו מן הפרק החמישי | |
|
|
והחלק הראשון אניח שהיה דבר אחד |
|
|
והשני צריך שיהיה עשרה פחות דבר אחד |
| ואזכירך שצריך להשיב כל דבר אל שרש | |
|
עתה תשיב ג' אל שרש ועושה ט' |
|
וכן תשיב דבר אחד אל שרש ועושה א' צינסו |
|
|
אח"כ תכפול ט' בא' צינסו ועושה ט' צינסי וזה לך בעד אחד מהחלקים |
|
|
אח"כ כמו שהשבותה למעלה השב עשרה פחות דבר אחד אל שרש ועושה שרש ק' פחות כ' דברים ויותר א' צינסו |
| וזה תכפול בשרש ח' ועושה שורש ת"ת פחות ק"ס דברים ויותר ח' צינסי אשר הם שוים אל ט' צינסי | |
|
| |
|
|
ועתה תשוה החלקי' ותן לכל אחד ק"ס דברי' |
|
|
ויהיה לך ט' צינסי וק"ס דברים שוים לת"ת מספרי' וח' צינסי |
|
|
תשוה עוד והוצא ח' צינסי מט' צינסי |
|
|
ויהיה לך א' צינסו וק"ס דברי' שוים אל ת"ת מספרי' |
| חלק עתה על הצינסי ויגיע לך ק"ס דברי' ות"ת מספרי' | |
|
תחצה הדברים ויהיה לך פ' |
|
תכפלם בעצמם ועושה ו' אלפים ות' |
|
תחברם אל המספר שהוא ת"ת ויעשה ז' אלפים ור' |
| ושרש ז' אלפים ור' פחות מחצית הדברים שהוא פ' היה אחד מהחלקי' שהוכה בשרש ט' | |
|
| |
| והחלק האחר היה הנשאר עד עשרה אשר הוכפל בשרש ח' וזה החלק יגיע להיות צ' פחות שרש ז' אלפים ור' | |
| ונעשה בעבור הפרק החמישי | |
| עוד בעבור הפרק החמישי החשבון האמור רצוני להניחו אל הפרק החמישי | |
|
|
ותניח כי היה אחד מהחלקים דבר אחד |
|
|
והאחר היה עשרה פחות דבר אחד |
|
תשיב כל החלקים אל שרש |
|
|
ויהיה לך א' צינסו לחלק אחד |
|
|
וק' מספרים פחות כ' דברים וא' צינסו לחלק האחר |
|
|
ועתה תכפול החלק הראשון שהוא א' צינסו בח' ועושה ח' צינסי וזהו לך לחלק אחד |
| תכפול השני בט' ויהיה לך תת"ק מספרי' פחות ק"פ דברים ויותר ט' צינסו שהם שוים לח' צינסי | |
|
| |
|
|
תשוה החלקים ותן ק"פ דברים לכל אחד מן החלקים |
|
|
ויהיה לך ח' צינסי וק"פ דברים שוים אל תת"ק מספרים וט' צינסי |
|
|
השוה עוד החלקי' והוציא ח' צינסי מט' צינסי |
|
|
ויהיה לך ק"פ דברים שוים לתת"ק מספרי' וא' צינסו |
| תחלק על הצינסו | |
|
|
ויהיה לך ק"פ דברים שוים אל תת"ק מספרים וא' צינסו |
|
תחצה הדברים ויהיה לך צ' |
|
תכם בעצמם ויהיה לך ח' אלפים וק' |
|
תוציא המספרים וישאר לך ז' אלפים ור' |
| ומשרש זה תוציא מחצית הדברי' וככה ישוה הדבר | |
|
| |
| ואתה הנחת שהחלק הראשון היה דבר אחד א"כ היה צ' פחות שרש ז' אלפים ור' | |
|
|
והחלק האחר היה הנשאר עד עשרה שהוא שרש ז' אלפים ור' פחות פ' |
| ונעשה עם הפרק החמישי | |

|
עוד בעבור הפרק האמור עשה לי מי"ו שני חלקים שכאשר הוכה האחד באחר יעשה מ"ח |
|
|
תניח שאחד החלקים היה א' דבר |
|
|
והיה האחר בהכרח י"ו פחות דבר אחד |
|
|
עתה תכה דבר אחד בי"ו פחות דבר אחד ועושה י"ו דברים פחות א' צינסו שהם שוים אל מ"ח |
|
|
תשוה א"כ החלקי' ויהיה לך י"ו דברים שוים למ"ח מספרי' וא' צינסו |
|
תחצה הדברי' ויהיה לך ח' דברים |
|
תכם בעצמם ויעשה ס"ד |
|
הוצא מזה המספר שהוא מ"ח ישאר י"ו |
| ותוציא שרש הי"ו ממחצית הדברים ויהיה לך לחלק האחד ח' פחות שרש י"ו | |
| או אם תרצה אמור השרש מי"ו תחבר על מחצית הדברים וככה ישוה הדבר | |
|
| |
| ואתה הנחת דבר אחד | |
|
|
א"כ היה ח' פחות שרש י"ו שהוא ד' וישאר ד' |
|
|
או אם תרצה אמור היה שרש י"ו שהוא ד' ויותר ח' שיבא להיות י"ב |
| וכן יגיע באופן האחד כמו באחר כי אם תשים החלק הראשון י"ב האחר יהיה ד' | |
| וכן הוא טבע זה הפרק החמשי | |
Chapter Six |
הפרק הששי |
| Its nature is when things and numbers are equal to squares.
|
וטבעו כאשר הדברים והמספרים יהיו שוים אל הצינסי |
| The whole equation should be divided by the number of the squares. | צריך לחלק כל ההשואה על כמות הצינסי |
| Then, the things should be divided into halves. | ואח"כ לחלק הדברים לחצאים |
| One of the halves is multiplied by itself | ולכפול אחד מהחצאים בעצמו |
| Add this product to the number. | ואותה ההכאה תחבר על המספרים |
| The root of this sum plus half [the number of] the things is equal to the thing.
|
ושרש אותו הסך ויותר המחצית האחד מהדברים יהיה שוה הדבר |
|
המשל תמצא לי מספר אחד שכאשר הוכה בעשרה ואותה ההכאה תחובר אל י"ב יעשה כמו המספר ההוא מוכה בעצמו |
|
תעשה כמו שרוצה זה הכלל שלו |
|
תניח שהמספר הוא דבר אחד |
|
וכאשר הוכה בעשרה יעלה עשרה דברים |
|
עתה תחבר אל זאת ההכאה י"ב ויהיה לך עשרה דברי' וי"ב מספרים אשר צריך שיהיו שוים אל א' צינסו דהיינו דבר אחד שהיה המספר מוכה בעצמו |
|
עתה תרדוף כפי הכלל האמור למעלה |
|
שיבא א' צינסו שוה לעשרה דברים וי"ב מספרי' |
|
עתה תחצה המספרי' הדברים ויגיע ה' |
|
תכם בעצמם ויהיה כ"ה |
|
וזאת ההכאה תחבר על י"ב ויהיה לך ל"ז |
|
ושר' ל"ז ושורש המחצית האחר מהדברי' יבא להיות הדבר דהיינו המספר |
|
א"כ יהיה לך שהמספר יהיה ה' ושרש ל"ז |
|
| |
| It is solved according to chapter six. | ונעשה בעבור הפרק הששי |
| Another calculation given for the said chapter: | עוד זה החשבון יושם בעבור הפרק האמור |
|
תמצא לי מספר אחד שכאשר נפיל ממנו שלישיתו ורביעיתו ומה שישאר יכפל בעצמו יעשה המספר ואחד יותר |
|
עתה תחלק על הצינסי ויהיה לך א' צינסו וזהו כאלו תאמר תחלק כ"ה חלקים מקמ"ד בצינסו על כ"ה חלקים מקמ"ד ויגיע לך א' שהוא א' צינסו |
|
עתה תחלק דבר אחד על כ"ה חלקים מקמ"ד ויגיע לך ה' וי"ט חלקי' מכ"ה בדראמ' |
|
|
וכן יהיה לך ה' דברים וי"ט חלקי' מכ"ה בדבר וה' דראמ' וי"ט חלקים מכ"ה בדרמ' תשוה לא' צינסו |
| תחצה הדברים שהם ב' וכ"ב חלקי' מכ"ה ותכם בעצמם ועושים ח' וקפ"ד חלקים מתרכ"ה | |
|
| |
| Solved according to [chapter] six. | וכך היה ונעשה בעד הששי |
Complex Equations |
|
| Know that here begin other chapters that are derived from the six chapters written above, in which squares, cubes, and squares of squares are specified, as you will see further in this book explained well. | דע כי בכאן מתחיל פרקים אחרים אשר הם מוצאים מן הו' פרקים הכתובים למעלה ובהם יהיו נקובים צינסי ומעוקבים וצינסי מצינסי כמו שתראה בהמשך זה הספר מבואר היטב |
Chapter Seven |
הפרק השביעי |
| When cubes are equal to numbers:
|
כאשר המעוקבים יהיו שוים אל המספרי' |
| The numbers should be divided by the cubes | צריך לחלק המספרים על המעוקבי' |
| The cube root of the result is equal to the thing.
|
והעולה מזה הנה שרשו המעוקב יבא לשוות הדבר |
|
תמצא לי שני מספרים שיהיה האחד חלק מהאחר כמו שהוא ג' מד' וכשיוכה הקטן בעצמו והיוצא תכה בגדול יעשה רפ"ח |
|
זהו הכלל שלו |
|
תניח אחד מהמספרים יהיה ג' דברים והאחר יהיה ד' דברים |
|
תכה הקטון שהוא ג' בעצמו ועולה ט' צינסי |
|
ואח"כ תכה ט' צינסי במספר הגדול שהוא ד' דברים ועולה ל"ו מעוקבים שהם שוים אל רפ"ח |
|
עתה תרדוף כפי הכלל האמור |
|
והוא שתחלק רפ"ח על ל"ו שעולה מזה ח' וככה יבא להיות המעוקב |
|
והדבר יבא להיות שרשו המעוקב שהוא שרש מעוקב מח' שהוא ב' |
|
ואתה הנחת שהמספר ראשון או אמור הקטון היה ג' דברים והדבר יבא להיות ב' א"כ ג' דברים יהיו ו' וככה היה המספר ראשון |
|
והמספר השני היה ד' דברי' והדבר הוא ב' א"כ ד' דברים יהיו ח' וככה הוא המספר השני |
| If the number of the cubes has no cube root, and you wish to know how much are the first and the second numbers that are 3 things and 4 things: | ואם המספר אשר יבא להיות המעוקב לא יהיה לו שרש מעוקב ורצית לדעת כמו יבא להיות או לשוות המספר ראשון והשני שהם ג' דברים וד' דברי' |
|
הנך צריך להכות ג' בשרש מעוקב מח' בזה האופן |
|
השב ג' לשרש מעוקב ויהיה לך שרש מעוקב מכ"ז |
|
וזה תכה בשרש מעוקב מח' ויעשה שרש מעוקב מרי"ו |
|
ושרש מעוקב מרי"ו שהוא ו' יבא לשוות המספר הראשון |
|
ואח"כ המספר האחר שהיה ד' דברים |
|
השיבנו אל שרש מעוקב ויהיה לך שרש מעוקב מס"ד |
|
וזה תכה בשרש מעוקב מח' שיבא שרש מעוקב מתקי"ב שהוא ח' וככה הוא המספר השני |
Chapter 8 |
הפרק הח' |
| When cubes are equal to things:
|
כאשר המעוקבים יהיו שוים אל הדברי' |
| The things should be divided by the cubes. | צריך לחלק הדברי' על המעוקבי' |
| The square root of the result is equal to the thing.
|
והעולה מזה הנה שרשו המרובע שוה הדבר |
|
תמצא לי שני מספרים שהאחד יהיה כפל השני וכשהוכה הקטון בעצמו ואותה ההכאה תוכה במספר השני יעשה כמו השני רצו' הגדול מוכה על ט' |
|
זהו הכלל שלו |
|
תניח שהמספר הראשון יהיה דבר אחד א"כ והשני צריך להיות ב' דברים א"כ יהיה כפלו |
|
עתה תכה המספר הקטון בעצמו שהוא דבר אחד ועלה א' צינסו |
|
וזאת ההכאה שהוא א' צינסו אכנה במספר השני שהוא ב' דברים שעולה ב' מעוקבי' ושמור זה לחלק אחד מן ההשואה |
|
ועתה תכה המספר השני שהוא ב' דברים בט' ועולה י"ח דברים וככה יהיה החלק האחר מההשואה |
|
ויהיה לך ב' מעוקבים שוים אל י"ח דברים |
|
עתה תרדוף כפי הכלל האמור |
|
תחלק הדברים על המעוקבים שהוא י"ח על ב' ויעלה מזה ט' |
|
ושרש זה הט' יבא להיות שוה הדבר וזה השרש יהיה ג' וככה הוא המספר הראשון |
|
והשני צריך להיות פי שנים מהראשון אם כן יבא להיות ששה |
| If the number has no root, meaning the quotient of the number of the things divided by the number of the cubes: | ואם המספר לא היה לו שרש רצוני העולה בחלוק הדברי' עם המעוקבים |
| Multiply the result by 4 and the root of this product is the second number, because it should be its double.
|
תכה מה שעולה בד' ושרש אותו הסך יבא להיות המספר השני מפני שצריך להיות כפלו |
| If it is its thrice, it should be multiplied by 9.
|
ואם יהיה ג' דמיוניו היה צריך לכפלו או להכותו על ט' |
| And so on, the numbers should be converted to roots as you saw above. | וכן לדמיוני אלו המספרי' בהשיב המספרים אל שרשים כאשר הראית למעלה |
| Know that this chapter is of the nature of the second: | ודע כי זה הפרק הוא מטבע השני כי ככה שוה |
| When cubes are equal to things it is the same as when squares are equal to numbers.
|
כאשר המעוקבים יהיו שוים אל הדברים כמו שהוא כאשר הצינסי יהיו שוים אל המספרים |
| The reason is that when dividing all the equation by things: | והסבה בזה היא כי לחלוק או לבקע ההשואה על הדברים |
| The cubese become squares.
|
המעוקבי' יצאו צינסי |
| The things become numbers.
|
והדברים יצאו מספרים |
| Since, when multiplying a thing by a square the result is a cube.
|
מפני כי להכות דבר בצינסו יצא מעוקב |
| When multiplying a number by a thing the result is thing[s].
|
ובהכות מספר בדבר יגיע דבר |
| Therefore, when dividing cubes by things, the result is squares.
|
א"כ לחלק המעוקבים על דברי' יעלה ממנו צינסי |
| When dividing things by things, the result is numbers.
|
ולחלוק דברי' על דברי' יגיעו מספרים |
| Thus, it is returned to the second chapter. | ויהיה כבר שב אל הפרק השני |
Chapter Nine |
הפרק התשיעי |
| When cubes are equal to squares:
|
כאשר המעוקבי' יהיו שוים אל הצינסי |
| The squares should be divided by the cubes and the result is equal to the thing.
|
צריך לחלק הצינסי על המעוקבים והעולה ממנו שוה הדבר |
|
תמצא לי שני מספרים שיהיה חלק האחד מהאחר כמו שהוא ג' מד' וכאשר הוכה הראשון בשני ואותה ההכאה תוכה על מקובץ שני המספרי' יחד יעשה כמו הכאת הגדול בעצמו |
|
זהו הכלל שלו |
|
תניח שא' מהמספרי' יהיה ג' דברים והשני ד' דברי' |
|
עתה תכה ג' דברי' בד' דברים שעולה י"ב צינסי |
|
וזאת ההכאה תכה בשני המספרים מקובצים יחד שהם ז' דברים שעולים פ"ד מעוקבי' ושמרם |
|
עתה תכה הגדול בעצמו שהוא ד' דברים שעולה י"ו צינסי וזאת ההכאה היא שוה לפ"ד מעוקבי' |
|
עתה תרדוף כפי הכלל האמור קודם |
|
תחלק הצינסי על המעוקבים שהם י"ו צינסי על פ"ד מעוקבים ויעלה ד' חלקי' מכ"א וככה שוה הדבר |
|
ואם רצינו לדעת כמה יבא להיות החלק הראשון אתה הנחת שהחלק הראשון היה ג' דברים והדבר הוא ד' חלקים מכ"א א"כ ג' דברים יהיו י"ב חלקים מכ"א שיבא להיות ד' חלקים מז' וככה הוא המספר הראשון |
|
והשני אתה הנחת שהיה ד' דברים והדבר שוה ד' חלקי' מכ"א א"כ ד' דברים יהיו י"ו חלקים מכ"א וככה הוא המספר השני |
| Know that this equation can be returned to the first chapter. | ודע כי זאת ההשואה אפש' להשיבה אל הפרק הראשון |
| The reason is that when we divide the whole equation by the squares: | וסבת זה היא זאת נחלק או בבקע כל ההשואה על הצינסי |
| The cubes become things.
|
המעוקבי' ישובו אל דברים |
| The squares become numbers.
|
והצינסי ישובו אל מספרי' |
Chapter Ten |
הפרק העשירי |
| When cubes are equal to squares of squares:
|
כאשר המעוקבים יהיו שוים אל הצינסי מצינסי |
| The cubes should be divided by the squares of squares and the result is equal to the thing.
|
צריך לחלק המעוקבים על הצינסי מצינסי ומה שיעלה מזה ככה שוה הדבר |
|
תמצא לי שני מספרים מתיחסים שיהיה חלק הראשון מהשני כמו שהוא ד' מה' וכשיוכה הראשון בשני ואותה ההכאה תוכה בעצמה ויעשה כמו שעושה הראשון מוכה בעצמו ואותה ההכאה תוכה במספר השני |
|
תעשה כמו שרוצה כללו זה |
|
תניח שהראשון יהיה ד' דברים והשני יהיה ה' דברי' |
|
עתה תכה ד' דברים בה' דברי' שעולה כ' צינסי |
|
וזאת ההכאה מכ' צינסי תכה בעצמה שעולה ת' צינסי מצינסי ושמור |
|
עתה תכה החלק הראשון בעצמו שהוא ד' דברים ועולה י"ו צינסי |
|
ואלו הי"ו צינסי תכה במספר השני שהוא ה' דברי' ויעלה פ' מעוקבי' וזאת ההכא' שוה אל ת' צינסי מצינסי |
|
ועתה תרדוף כפי הכלל האמור למעלה |
|
תחלק המעוקבי' על הצינסי מצינסי שהוא פ' על ת' ויעלה ה' וככה שוה הדבר |
|
ואתה הנחת שהמספר הראשון היה ד' דברי' והדבר הוא א' חומש א"כ ד' דברים יהיו ד' חלקי' מה' וככה הוא המספר הראשון |
|
והמספר השני היה ה' דברי' וה' דברים שוים ה' חמישיות שהם אחד וככה הוא המספר השני |
| Know that this equation can be returned to the first chapter: | ודע כי זאת ההשואה אפש' להשיבה אל הפרק הראשון |
| Because, when the cubes are divided by cubes, they become numbers.
|
מפני כי בבקעו המעוקבי' על המעוקבים יבאו להיות מספרים |
| The squares of squares become things.
|
והצינסי מצינסי יבאו להיות דברים |
Chapter 11 |
הפרק הי"א |
| When squares of squares are equal to numbers:
|
כאשר צינסי מצינסי יהיו שוים אל מספרים |
| The numbers should be divided by the squares of squares. | צריך לחלק המספרים על הצינסי מצינסי |
| Extract a root of a root of the result and this is the thing.
|
ומהעולה מזה תקח שרש שרשו והוא יהיה הדבר |
|
תמצא לי מספר אחד שכשיוכה בשני שלישיו ועולה שני שלישי צינסו והעולה יוכה בעצמו
יעשה ל"ו |
| Its rule: | זהו הכלל שלו |
|
תניח שהמספר יהיה דבר אחד |
|
|
עתה תכה דבר אחד בשני שלישיו ועולה שני שלישי צינסו |
|
|
ועתה תכה ב' שלישי צינסו על עצמם |
|
|
ועולה ד' תשיעיות מצינסו דצינסו שהם שוים ל"ו מספרי' |
|
עתה תרדוף כפי הכלל האמור למעלה |
|
|
תחלק המספרים על הצינסי מצינסי דהיינו ל"ו על ד' תשיעיות ויבא מזה פ"א וככה יבא להיות הצינסי מצינסי |
|
|
ושרש השרש מפ"א יבא להיות הדבר דהיינו המספר הנשאל אשר הנחת היותו דבר אחד וזה שרש השרש יגיע להיות ג' וככה הוא המספר |
| This is almost similar to the nature of the second chapter, except that here the root of the root is extracted while in the second chapter only one root is extracted. | ודע כי זה כמעט דומה לטבע הפרק השני רק שלוקח שרש השרש והשני לוקח שרש אחד לבד |
Chapter 12 |
הפרק הי"ב |
| עוד רודף באופן אחר דהיינו | |
| When squares of squares are equal to things:
|
כאשר הצינסי מצינסי יהיו שוים אל הדברי' |
| The things should be divided by the squares of squares. | צריך לחלק הדברים על הצינסי מצינסי |
| The cube root of the result is equal to the thing.
|
והעולה מזה הנה שרשו המעוקב יהיה שוה הדבר |
|
תמצא לי מספר אחד שכאשר הוכה בעצמו ומה שיעלה יוכה בשני שלישיו יעשה כמו ו' דמיוני המספר האמור אשאל כמה יהיה המספר האמור |
| Following its rule: | תעשה כמו שאומ' זה הכלל שלו |
|
תניח שהמספר יהיה דבר אחד |
|
|
ותכהו בעצמו ויהיה א' צינסו |
|
|
וזה א' צינסו תכהו בשלשת חלקיו רצוני בג' רביעי צינסו ועולה ג' רביעי צינסו מצינסו ושמרם |
|
|
ועתה תכה המספר שהוא ו' בדבר אחד ועושה ו' דברים |
|
|
ואלו ו' דברים הם שוים אל ג' רביעי' צינסו מצינסו |
|
עתה תרדוף כפי הכלל האמור למעלה |
|
|
והוא שאתה צריך לחלק הדברים על הצינסו דהיינו ו' בג' רביעי' ויגיע לך ח' ושרש המעוקב מח' יבא להיות הדבר שהוא המספר אשר הנחת היותו הדבר |
| The [equation in this] chapter can be restored to chapter seven: | ודע כי זה הפרק אפש' להשיבו אל הפרק השביעי |
|
|
מפני כי לבקע צינסו מצינס' על דבר יצא ממנו מעוקב |
|
|
ודבר על דבר יצא ממנו מספר |
Chapter 13 |
הפרק הי"ג |
| When squares of squares are equal to squares of squares:
|
עוד תדע כאשר הצינסי מצינסי יהיו שוים אל הצינסי |
| The the whole equation, i.e. the squares should be divided by the squares of squares. | צריך לחלק כל ההשואה דהיינו הצינסי על הצינסי מצינסי |
| The square root of the result is equal to the thing.
|
והעולה מזה שרשו המרובע ישוה הדבר |
|
תמצא לי שני מספרים שיהיה חלק הראשון מהשני כמו שהוא ג' מה' וכאשר הוכה הקטון בגדול ומה שיעלה יוכה בעצמו יעשה כמו הכאת הגדול בעצמו |
| Its rule: | זהו כללו |
|
תניח שהמספר הראשון יהיה ג' דברים |
|
והאחר ה' דברים |
|
|
עתה תכה הראשון בשני שזהו ג' דברים בה' דברים עושים ט"ו צינסי |
|
|
וזאת ההכאה רצוני ט"ו צינסי תכה בעצמה ועולה רכ"ה צינסי מצינסי ושמרם |
|
|
עתה תכה החלק הגדול בעצמו שהוא ה' דברים |
|
|
ועולה כ"ה צינסי שהם שוים אל רכ"ה צינסי |
|
עתה תרדוף כפי הכלל האמור למעלה |
|
|
תחלק הצינס' על הצינסי מצינסי דהיינו כ"ה על רכ"ה שעולה מזה א' תשיעית וכן שוה הצינסו |
|
|
ושרש א' תשיעית יבא להיות הדבר והשרש הזה יבא להיות א' שלישי' וכן הוא הדבר |
|
|
ואתה הנחת המספר הראשון היותו ג' דברי' והדבר הוא א' שלישי א"כ ג' דברי' יהיו אחד שלם וכן הוא המספר הראשון |
|
|
והמספר השני הונח היותו ה' דברים והדבר הוא א' שלישי א"כ יהיו ה' שלישים שהם א' וב' שלישי' וכן הוא המספר השני |
If  has no root: has no root:
|
ואם המספרי' המגיעי' לך בחלוק הצינסי על הצינסי מצינסי {לא היה להם שרש |
|
|
ובקשת לדעת כמה הוא ג' דברים תכה ג' בעצמו עושה ט' ולכן תכה מה שעלה שהוא א' תשיעי' בט' ועושה א' שלם |
|
|
והמספר השני אשר הונח ה' דברי' תכה ה' בשרש א' תשיעית שעולה שרש כ"ה תשיעיות שהוא ה' שלישיו' |
| This equation can be restored to chapter two, by dividing all the equation by a square: | ודע כי זאת ההשואה אפש' להשיבה אל הפרק השני מפני כי בחלק ההשואה על הצינסי |
|
|
מצינסי יצא צינסי |
|
|
והצינסי מצינסי יצאו מספרים |
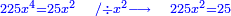 which is restored to chapter two. which is restored to chapter two.
|
א"כ רכ"ה צינסי יבאו שוים אל כ"ה צינסי סקיסאנדי שהוא כ"ה מספרים ויבא מושב אל הפרק השני רכ"ה צינסי שוים אל כ"ה מספרים |
Chapter 14 |
פרק י"ד |
| When cubes and squares are equal to things:
|
כאשר המעוקבים והצינסי יהיו שוים אל הדברי' |
| The whole equation should be divided by the number of the cubes. | צריך לחלק כל ההשואה על כמות המעוקבים |
| Then, [the number of] the squares should be halved, i.e. in two equal parts. | ואח"כ לחלק הצינסי לחצי רצו' לשני חלקים שוים |
| Multiply one of the halves by itself. | ואחד מאותם החצאים תכה בעצמו |
| Add the product to [the number of] the things. | ואותה ההכאה תחבר אל הדברים |
| The root of this sum minus the other half of [the number of] the squares is equal to the thing and the thing is a root of the square.
|
ושרש זה הסך פחות המחצית האחר מהצינסי יבא לשוות הדבר והדבר יבא להיות שרש ממה שהוא הצינסו |
|
והנה המשל תמצא לי שלשה מספרים שיהיה חלק הראשון מהשני כמו שהוא ב' מג' והשני יהיה מהשלישי כמו שהוא ג' בד' |
| Its rule: | זהו הכלל שלו |
|
תניח שהמספר הראשון היה ב' דברים |
|
והשני ג' דברי' |
|
והשלישי ד' דברי' |
|
|
עתה תכה המספר הראשון בעצמו רצוני ב' דברים ועולה ד' צינסי |
|
|
עתה תכה זאת ההכאה שהיא ד' צינסי במספר הראשון שהיא ב' דברים ועולה ח' מעוקבים ושמרם |
|
|
אח"כ תכה המספר השני בעצמו שעולה ט' צינסי |
|
|
וחברם על הח' מעוקבים אשר שמרת ויהיה לך ח' מעוקבי' וט' צינסי ושמור זה הסך בעד אחד מהחלקים |
|
|
אח"כ תכה המספר השלישי דהיינו ד' דברי' בי"ב וחצי ועולה נ' דברי' שהם החלק האחר מההשואה |
|
|
א"כ ח' מעוקבי' וט' צינסי הם שוים אל נ' דברים |
|
עתה תרדוף כפי הכלל האמור למעלה |
|
והוא שאתה צריך לחלק כל דבר בכמו מה שהם המעוקבים רצוני כל ההשואה על ח' |
|
|
ויעלה לך א' מעוקב וא' צינסו וא' שמינית שוים אל ו' דברים ורביע |
| עתה צריך אתה לחלק הצינסי לחצי ויעלה ט' חלקים מי"ו | |
| ואלו הט' חלקים מי"ו תכם בעצמם ויעלה פ"א חלקים מרנ"ו | |
| וזאת ההכאה תחבר על הדברי' שהם ו' ורביע ויהיה לך ו' וקמ"ה חלקים מרנ"ו | |
| ושרש אלו הו' וקמ"ה חלקים מרנ"ו פחות המחצית האחר מהצינסי שאומ' פחות ט' חלקים מי"ו יבא לשוות הדבר | |
| ושרש זה הוא ב' וט' חלקים מי"ו | |
| ומזה צריך להוציא המחצית האחד מהצינסי שהוא ט' חלקים מי"ו וישאר ב' וכן שוה הדבר | |
|
| |
|
|
ואתה הנחת היות המספר הראשון ב' דברי' א"כ יבא להיות המספר הראשון ד' |
|
|
והמספר השני שמת היותו ג' דברי' שיבא להיות ו' |
|
|
והשלישי הנחת היותו ד' דברים א"כ היה ח' |
| These are the numbers required above. | וכן יבאו להיות המספרי' המבוקשים למעלה |
The [equation in] this chapter can be restored to chapter 4, by dividing all the equation by a thing 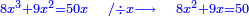
|
ודע כי זה הפרק אפש' להביאו אל הפרק הרביעי מפני כי להבקיע כל ההשואה על דבר יגיע ח' צינסי וט' דברים שוים אל נ' דברים |
which can be divided then by the number of the squares 
|
או נאמר א' צינסו וא' שמינית וא' דבר שוים לו' דברים ורביע בהיות נחלק כפי מה שהם הצינסי |
Chapter 15 |
פרק ט"ו |
| When cubes and things are equal to squares:
|
כאשר המעוקבים והדברים יהיו שוים אל הצינסי |
| The whole equation should be divided by the numbers of cubes. | צריך לחלק כל ההשואה על כמו שהם המעוקבים |
| Then, [the number of] the squares should be halved. | ואח"כ לחלק הצינסי לחצאים |
| Multiply one of the halves by itself. | ואחד מהחצאי' תכהו בעצמו |
| Subtract the number of the things from this product. | ומאותה ההכאה תוציא כמות הדברים |
| We extract the root of the remainder. | ומן הנשאר נקח שרשו |
| We add it to the other half of [the number of] the squares and this is equal to the thing.
|
ונוסיפהו על המחצית האחר מהצינסי וככה ישוה הדבר |
|
|
ופעמים מה תצטרך לומ' שהדבר שוה מחצית הצינסי פחות השרש מאשר הנשאר |
|
|
ופעמים מה יהיה אפשר לענות שהדבר ישוה מחצית הצינסי ויותר שרש הנשאר |
| According to the way of the fifth chapter. | או פחות שרש הנשאר כפי האופן מהפרק החמישי |
|
והנני עושה לך מזה המשל עשה לי מחלקים מעשרה שני חלקים באופן שכאשר יוכה ההבדל שבין האחד אל האחר בעצמו וזאת ההכאה תוכה בחלק הגדול יעשה כמו הכאת החלק הגדול בעשרים
ורביע |
| Its rule: | זהו כללו |
|
תניח שאחד מהחלקי' יהיה דבר אחד |
|
והאחר יבא להיות עשרה פחות דבר אחד |
|
|
עתה תקח ההבדל שהוא בין זה לזה שיבא להיות ב' דברים פחות עשרה |
| When we want to restore it to the first aforementioned answer of this chapter. | ברצותנו להשיבה אל זה הפרק בעד התשובה הראשונה האמורה למעלה |
|
|
עתה תכה זה ההבדל בעצמו דהיינו ב' דברים פחות עשרה ועולה ד' צינסי ועשרה דברים פחות מ' דברים |
|
|
וזאת ההכאה תכה עוד בחלק הגדול אשר אני עושה שהוא דבר אחד כדי להשיב החשבון אל זה הפרק ויעלה ד' מעוקבי' וק' דברים פחות מ' צינסו ושמור זה בעבור אחד מהחלקי' |
|
|
עתה תכה החלק הגדול אשר עשינוהו היותו דבר אחד בכ' ורביע ויעלה כ' דברי' ורביע וזה יהיה החלק השני מההשואה |
|
|
ויהיה לך ד' מעוקבי' וק' דברי' פחות מ' צינסי שוים לכ' דברים ורביע |
|
|
עתה תחבר מ' צינסי שהם גורעים לאחד מהחלקים ובדומה לזה תחבר עוד אל החלק האחר |
| As said above that instead subtracting one needs to add, so one needs to add to one side the same as the other. | כאשר נאמ' לך לפנים שבמקום הגורע צריך להוסיף וכן צריך להוסיף על החלק האחד כמו אל האחר |
| Since you added to the one as to the other, you need to subtract the smaller quantity of the things from each side. | ואחר שהוספת על האחד כמו לאחר צריך אתה להוציא הכמות הקטון מהדברי' מכל אחד מהחלקי' |
|
|
וישאר לך ד' מעוקבי' וע"ט דברי' וג' רביעים שוים אל מ' צינסי |
|
עתה תחלק כל ההשואה הזאת השניה כאמור למעלה דהיינו לחלוק בכמות המעוקבים שיבא לחלוק על ד' |
|
|
ויבא א' מעוקב וי"ט דברים וט"ו חלקים מי"ו שוים אל עשרה צינסי |
| עתה תחלק הצינסי לחצי ויעלה ה' | |
| ואלו הה' תכם בעצמם ועולה כ"ה | |
| ומאלו הכ"ה תוציא כמות שהם י"ט וט"ו חלקים מי"ו וישאר ה' וחלק א' מי"ו | |
| ושרש ה' וא' חלק מי"ו שהוא ב' ורביע תוסיף על המחצית האחד מהצינסי שהוא על הה' ויהיה לך שהדבר יבא להיות ז' ורביע | |
|
| |
|
|
או אם תרצה אמור ה' ויותר שרש מן ה' וחלק א' מי"ו כפי מה שנתן הפרק האמור וכן יבא להיות החלק הגדול |
|
|
והאחר יבא להיות הנשאר עד עשרה שהוא ב' וג' רביעים |
|
|
או אם תרצה אמור ה' פחות שרש ה' וחלק מי"ו והוא החלק הקטן |
| This equation is restored to chapter 5, by dividing all the equation by a thing, which yields the answer by the first way so that the thing is a number plus a root
|
ודע כי זאת ההשואה שהיא מעוקבים ודברי' שוים לצינסי ישוב אל הפרק החמישי בחלוק על דבר כל ההשואה ותעשה לך התשובה באופן הראשון היות הדבר מספר ויותר שרש |
| Another calculation whose answer is by the second way of this chapter, which is a number minus a root. | עוד רצוני לתת לך חשבון אחר שתשובתו תהיה מזה הפרק באופן השני שהוא מספר פחות שרש |
| I will give you another question for chapter 15: | עוד אניח לך שאלה אחרת בעד פרק ט"ו האמור |
|
עשה לי מעשרה שני חלקים באופן שכאשר יוכה ההבדל שבין שני החלקים בעצמו ואותה ההכאה תכה בחלק הקטון יעשה כמו הכאת החלק הקטון בכ' ורביע אשאל כמה יבא להיות כל אחד מהחלקים |
| Following its rule: | תעשה כמו שאומ' כללו |
|
תניח שאחד מהחלקי' יהיה דבר אחד |
|
והאחר יבא להיות עשרה פחות דבר אחד |
|
|
עתה תקח ההבדל שבין האחד אל האחר שהוא עשרה פחות ב' דברים |
| If we want to restore it now to the second answer of this chapter. | ואם רצינו להשיבה אל זה הפרק בתשובה השנית עתה |
|
|
תכה זה ההבדל בעצמו שהוא עשרה פחות ב' דברים ועולה ק' מספרים וד' צינסי פחות מ' דברים |
|
|
וזאת ההכאה מוכת על החלק הקטון אשר אנחנו עשינו היותו דבר אחד כדי להשיבו אל התשובה השנית ועולה השנית ועולה ק' דברים וד' מעוקבים פחות מ' צינסי ושמרם בעבור שאחד מהחלקים מההשואה |
|
|
עתה תכה החלק הקטון אשר עשינו היותו דבר אחד בכ' ורביע ויעלה כ' דברים ורביע וכן יהיה החלק השני מההשואה |
|
|
ויהיה לך מאה דברי' וד' מעוקבי' פחות מ' צינסי שוים אל כ' דברים ורביע |
|
|
עתה תוסיף מ' צינסי אשר פוחתים מאחד החלקים ואם תעשה כפי מה שהראית לפנים תוסיפם לחלק האחר גם כן ותוציא הכמות הקטון מהדברים מכל אחד מהחלקים שהוא כ' דברים ורביע |
|
|
וישאר ע"ט דברים וג' רביעים וד' מעוקבים שוים אל מ' צינסי |
|
עתה תחלק כל זאת ההשואה כפי מה שנאמ' לך לפנים דהיינו בכמו שהם המעוקבים שיבאו להחלק על ד' |
|
|
ויבא א' מעוקב וי"ט דברים וט"ו חלקים מי"ו שוי' אל עשרה צינסו |
| עתה תחלק הצינסי לחצאים ויבא מזה ה' | |
| ותכה ה' על עצמו ועושה מזה כ"ה | |
| ומאלו הכ"ה תוציא הדברי' שהם י"ט וט"ו חלקי' מי"ו וישאר ה' וחלק א' מי"ו | |
| ושרשם שהוא ב' ורביע תוציא מהחצי האחר מהצינסי שהוא ה' ויהיה לך היות הדבר ב' וג' רביעי' | |
|
| |
|
|
או אם תרצה תאמר ה' פחות שרש ה' וחלק מי"ו כפי מה שהפרק השיב לו וככה יבא להיות החלק הקטון |
|
|
והאחר יבא להיות הנשאר עד עשרה שהוא ז' ורביע |
|
|
או תאמ' ה' ושרש ה' וחלק מי"ו והוא החלק הגדול |
| The answer is done so that the root is subtracted in the thing as the stated method of the chapter. | ונעשתה התשובה להיות הדבר פחות שרש כפי אופן הפרק אשר אמרתי לך |
| I also want to show you how the chapter can assign the thing a number and a root and a number minus a root by that same calculation of chapter 15: | עוד רצוני להראות לך איך הפרק האמור אפש' ליתן הדבר מספר ושרש ומספר פחות שרש בחשבון אחד ממש עוד בעבור הפרק הט"ו האמור |
|
עשה לי מעשרה שני חלקים באופן שכאשר יוכה החלק הראשון בשני וההכאה ההיא תוכה בראשון יעשה כמו הכאת הראשון בכ"א אשאל כמה יבא להיות כל אחד מהחלקים האמורים |
|
|
זהו כללו תניח שהחלק הראשון יהיה דבר אחד |
|
|
והשני יבא להיות עשרה פחות דבר אחד |
|
|
ועולה עשרה דברים פחות א' צינסו |
|
|
וזאת ההכאה תכה עוד בחלק הראשון שהוא דבר אחד ועולה עשרה צינסי פחות א' מעוקב ושמור |
|
|
אח"כ תכה החלק הראשון שהוא דבר אחד בכ"א |
|
|
ועולה כ"א דברים והם שוים אל עשרה צינסי פחות א' מעוקב |
|
|
עתה תתן המעוקב שהוא פחות לכל אחד מהחלקים |
|
|
ויהיה לך א' מעוקב וכ"א דברים שוים אל עשרה צינסי |
|
עתה צריך אתה לחלק כל ההשואה בכמו שהם המעוקבים שהוא אחד |
|
ועולה מזה אותו בעצמו |
| עתה עליך לחלוק הצינסי לחצאים כמו שרוצה הכלל האמור ויעלה ה' | |
| ותכה ה' בעצמו ועולה כ"ה | |
| ומזה הכ"ה תוציא כמות הדברי' שהם כ"א וישאר ד' | |
| ושרש זה הד' תוסיף על מחצית כמות הצינסי דהינו על ה' או להוציא זה השרש חוצה מזה הה' | |
|
| |
| Since this calculation can be answered by addition and by subtraction, one can answer that the thing is 5 plus a root of 4, or say [that it is] 5 minus a root of 4, according to the third way of the chapter. | מפני כי זה החשבון תוכל לענות ביותר ובפחות א"כ תוכל לענות שהדבר הוא ה' ושרש ד' או תאמר ה' פחות שרש ד' כפי האופן השלישי מהפרק האמור |
Chapter 16 |
פרק י"ו |
| When squares and things are equal to cubes:
|
כאשר הצינסי והדברים יהיו שוים למעוקבים |
| The whole equation should be divided by the number of the cubes. | צריך לחלק כל ההשואה על כל כמות המעוקבים |
| Then, the number of the squares should be halved. | ואח"כ לחלק כמות הצינסי לחצאים |
| Multiply one of the halves by itself. | ולהכות אחד מהחצאים על עצמו |
| Add this product to the number of the things. | וזאת ההכאה תוסיף על כמות הדברים |
| Add the root of this sum to the other half of the number of the squares and it is equal to the thing, i.e. half of the number of the squares plus the root of this sum.
|
ושרש זה הסך תוסיף אל המחצית האחר מכמות הצינסי וככה יבא לשוות הדבר דהיינו מחצית כמות הצינסי ויותר שרש אותו הסך |
|
עשה לי החשבון תמצא לי מספר אחד שכאשר הוכה בעצמו ואותה ההכאה תוכה במספר ההוא יעשה כמו הכאת המספר האמור בי"ב ואח"כ להכות המספר האמור בעצמו ואותה ההכאה תוכה בעשרה ולחבר עם ההכאה שנעשתה בי"ב אשאל כמה יבא להיות המספר האמור |
|
|
זהו הכלל שלו תניח שהמספר יהיה דבר אחד |
|
|
ותכהו בעצמו ויהיה א' צינסו וזאת ההכאה תכה על המספר שהוא דבר אחד ועולה א' מעוקב ושמרהו בעבור חלק אחד מההשואות |
|
|
אח"כ תכה המספר האמור בי"ב דהיינו דבר אחד בי"ב ועולה י"ב דברים |
|
|
ואח"כ תשוב אל המספר האמור שהוא דבר אחד תכהו בעצמו ועלה א' צינסו וזה הצינסו תכהו בעשרה ועולה י' צינסו |
|
|
ואותם תחבר עם ההכאה העשויה בי"ב שהם י"ב דברים |
|
|
ויהיה לך עשרה צינסי וי"ב דברים שוים לא' מעוקב |
|
עתה תרדוף כפי הכלל האמור למעלה |
|
תחלק כל ההשואות כל כמות המעוקבים שהוא אחד |
|
ויעלה אותו בעצמו |
| עתה תחלק כמות הצינסי לחצאי' ויעלה ה' | |
| וזה הה' תכהו בעצמו ועולה כ"ה | |
| תוסיף אלו הכ"ה על כמות הדברים שהם י"ב ויהיה לך ל"ז | |
| ושרש אלו הל"ז תחבר אל המחצית האחר מהצינסי שהוא ה' ויהיה לך שהדבר יבא לשוות ה' ויותר שרש מל"ז וככה יבא להיות המספר האמור | |
|
| |
This equation can be restored to chapter 6, by dividing all the equation by a thing 
|
ודע כי זאת ההשואה אפש' להשיבה אל הפרק הששי בחלוק על דבר כל ההשואה ויעלה ח' צינסו שוה לו' דברים וי"ב מספרי' |
Chapter 17 |
פרק י"ז |
| When things are equal to a root of numbers:
|
כאשר הדברים יהיו שוים אל שרשי המספרים |
| The number [of the things] should be nultiplied by itself. | צריך להכות הכמות בעצמו |
| Then, the numbers should be divided by this product. | ולחלק המספרים על ההכאה ההיא |
| The root of the result is equal to the thing.
|
ושרש העולה מזה שוה הדבר |
|
תמצא לי שני מספרים שיהיה חלק האחד מהאחר כמו שהוא ב' מג' וכשיוכה כל אחד בארבעה ויקובצו אלו ההכאות יחד יעשו שרש ק' |
|
|
זהו הכלל שלו תניח שאחד מהמספרים יהיה ב' דברים |
|
|
והאחר יבא להיות שלשה דברים |
|
|
עתה תכה ב' דברים בד' ועולה ח' דברים ושמרם |
|
|
אח"כ תכה המספר האחד שהוא ג' דברים בד' ועולה י"ב דברים |
|
|
עתה תחבר ח' דברים עם י"ב דברים |
|
|
ועולים כ' דברים שהם שוים אל שרש ק' |
|
עתה תרדוף כפי הכלל האמור למעלה |
|
|
תכה כמות הדברים בעצמם שהם כ' דברים ויעלה ת' עתה תחלק כמות המספרים אשר נקבו שרש והם ק' ותחלק אלו הק' בהכאת כמות הדברים שהוא ת' שיבא א' רביע |
|
|
והמספר הראשון שמתו ב' דברים אשר יבא להיות שרש א' רביע דהיינו א' שלם וכן הוא המספר הראשון |
|
|
והשני הנחת היותו ג' דברים אשר יבא להיות ג' פעמים שרש א' ורביע שהוא א' וחצי וככה הוא המספר השני |
|
|
א"כ הראשון יבא להיות שרש האחד והשני יבא להיות שרש ב' ורביע |
| If one wishes to restore this equation to one of the six chapters, it can be restored to chapter two. | ואם רצית להשיב זאת ההשואה אל אחד מהו' פרקים דע שאפש' להשיבה אל הפרק השני |
Since 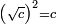
|
מפני כי שרש מספר מוכה בשרש מספר מוכה בעצמו אי פרודושא יעשה מספר |
and 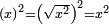
|
והדבר שהוא שרש מצינסו מוכה בעצמו עושה צינסו |
Therefore, 
|
וא"כ ת' צינסו הם שוים אל ק' מספרים |
Chapter 18 |
פרק י"ח |
| When numbers are equal to a root of things:
|
עוד כאשר המספרים יהיו שוים אל שרשי הדברים |
| The number should be nultiplied by itself. | צריך להכות המספר בעצמו |
| Then, this product should be divided by the number of the things that are the radicand; the result is equal to the thing.
|
ואותה ההכאה תחולק בכמות הדברים אשר נקבו בשמות והעולה מזה ישוה הדבר |
|
תמצא לי מספר אחד שכאשר יוכה בט' יהיה שרש העולה י"ב אשאל כמה יבא להיות המספר |
| Following its rule: | תעשה כמו שאומ' כללו זה |
|
תניח שהמספר יהיה דבר אחד |
|
|
ותכה דבר אחד בט' ועושה ט' דברים |
|
|
ושרש ט' דברי' הוא שוה אל י"ב |
|
עתה תרדוף כפי הכלל האמור למעלה |
|
|
תכה המספרים בעצמם שהוא י"ב על י"ב ועושה קמ"ד ותחלק על כמות הדברים אשר נקבו היות להם שרשים דהיינו על ט' ויעלה מזה י"ו |
The equation 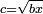 can be restored to chapter one in this way: can be restored to chapter one in this way:
|
ודע כי ההשואה הזאת דהיינו מספרים שוים אל שרשים דברים ישוב אל הפרק הראשון בזה האופן |
|
|
דהיינו תכה שרש ט' דברים בעצמו יעלה ט' דברי' |
|
|
וי"ב מספרי' שהם שרש מהכאה המוכה בעצמה עושה קמ"ד מספרי' |
Since 
|
מפני כי ככה שוה כאשר שרש הדבר שוה לשרש מספר כמו כשהדבר שוה למספר |
Because, when the roots of the things equal numbers, these [numbers] are also equal to the root of other numbers [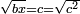 ]. ].
|
מפני כי בהיות שרשי הדברי' שוים אל מספרי' מה אותם הם ג"כ שרשים למספרי' אחרי' |
| Therefore, one should only convert those numbers to roots, and by this the things will be equal to numbers. | א"כ אין צורך רק להשיב אותם המספרי' אל שרשים ובאותו ההיות יהיה א"כ הדברי' עם המספרי' |
| Thus, it is restored to chapter one. | ויהיה מושב אל הפרק הראשון |
Chapter 19 |
פרק י"ט |
| When squares are equal to a root of numbers:
|
כאשר הצינסי יהיו שוים אל שרשי דראמי או מספרי' |
| The number of the squares should be nultiplied by itself. | צריך להכות כמות הצינסי בעצמם |
| Then, the numbers should be divided by the square, or by the product of [the number of] the squares. | ואח"כ לחלק הדראמי או המספרי' על המרובע או בהכאת הצינסי |
| The root of the root of the result is equal to the thing.
|
ושרש השרש מהעולה יבא לשוות הדבר |
|
תמצא לי שני מספרים שיהיה חלק האחד מהאחר כמו שהוא ב' מג' ואם יוכה האחד באחר יעשה שרש מי"ב |
| Following its rule: | תעשה כמו שאומ' זה הכלל שלו |
|
תניח שהאחד יהיה ב' דברים |
|
והאחר יהיה ג' |
|
|
עתה תכה האחד באחר שהוא ב' דברים בג' דברים ויעלה ו' צינסי |
|
|
ואלו הו' צינסי הם שוים אל שרש י"ב |
|
עתה תרדוף כפי הכלל האמור למעלה |
| תכה כמות הצינסי בעצמם והם ו' ועולה ל"ו | |
| ועתה תחלק המספרי' אשר נקבו להיות להם שרש והם י"ב על מרובע זה מהצינסי רצו' על ל"ו ויבא א' שליש | |
| ושרש השרש מזה שעלה שהיה א' שליש יבא להיות הדבר | |
|
| |
|
ואתה הנחת שהמספר הראשון היה ב' דברים א"כ תכה ב' על שרש משרש א' שליש ועולה שרש משרש ה' ושליש וככה יבא להיות המספר הראשון |
|
ואתה הנחת שהשני היה ג' דברי' והדבר הוא שרש השרש מא' ושליש א"כ תכה ג' בשרש השרש מא' ושליש |
The equation  can be restored to chapter 11 in this way: can be restored to chapter 11 in this way:
|
ודע כי זאת ההשואה רצוני שצינסי יהיו שוים אל שרשי המספרי' אפש' להשיבה אל הפרק הי"א בזה האופן |
|
תשיב ו' צינסי אל שרשים |

|
ויהיה לך שרשים מצינסי מצינסי שוים לשרשי' ממספרים ושוה כמו שהיו צינסי מצינסי שוים אל מספרי' |
| This way it is restored to chapter 11. | ותהיה מושבת בזה האופן אל הפרק הי"א |
Chapter 20 |
פרק כ' |
| When the numbers are equal to a root of squares:
|
עוד כאשר המספרי' יהיו שוים אל שרשי צינסי |
| The numbers should be multiplied by themselves. | צריך להכות המספרי' בעצמם |
| Then, this product should be divided by the number of the squares that are the radicand. | ולחלק ההכאה ההיא בכמות הצינסי הנקובים שהם להם שרש |
| The root of the result is equal to the thing.
|
ושרש העולה שוה הדבר |
|
והנה המשל תמצא לי שני מספרים שיהיה חלק אחד מהם לאחר כמו שהוא ב' אל ג' וכשיוכה הראשון בשלשה והשני בארבעה ואלו שתי ההכאות מחוברים יחד ויוכה בשרש ה' יעשה מ' |
|
זהו כללו |
|
תניח שהמספר הראשון יהיה ב' דברים והאחר יבא להיות ג' דברי' |
|
עתה תכה הראשון שהוא ב' דברים על ג' ועולה ו' דברי' ושמרם |
|
אח"כ תכה המספר השני שהוא ג' דברי' בד' ועולה י"ב דברי' |
|
ותחברם עם ו' דברי' אשר שמרת ויהיו לך י"ח דברי' |
|
עתה תכה י"ח דברים בשרש ה' |
|
זכור כי הנך צריך להשיב י"ח דברי' אל שרשים אשר יבא אל שרש שכ"ד צינסי |
|
אשר תכם בשרש ה' ויהיה לך שרש מאלף תר"כ צינסי שהם שוים אל מ' מספרים |
|
עתה תרדוף כפי הכלל האמור למעלה |
|
תכה המספרי' שהם מ' בעצמם ועולי' אלף ות"ר |
|
וחלק אלף ת"ר בכמות הצינסי אשר נקבו היות להם שרש שהם אלף תר"כ ויבא מזה פ' חלקים מפ"א |
|
ושרש פ' חלקים מפ"א יבא לשוות הדבר |
|
ואתה הנחת שהמספר הראשון היה ב' דברים א"כ תכפול ב' בשרש פ' חלקי' מפ"א ועולה שרש ג' וע"ז חלקים מפ"א וככה יבא להיות המספר הראשון |
|
והמספר השני הנחת היותו ג' דברי' א"כ תכה ג' בפ' חלקי' מפ"א ועולה שרש ח' וע"ב חלקים מפ"א וככה יבא להיות המספר השני |
| Know that this equation can be returned to the second chapter: | ודע כי זאת ההשואה אפש' להשיבה אל הפרק השני |
| Since a root of a square equals a root of a number is the same as a square equals a number.
|
מפני כי כך שוה שרש מצינסו שוה אל שרש מספר כמו צינסו שוה אל מספר |
|
|
וזה ראית באותו שחלקת שרש אלף ת"ר מספרי' בשרש אלף תר"כ צינסי |
| Know also that this equation can be returned to the first chapter: | ודע עוד כי זאת ההשואה אפש' להשיבה אל הפרק הראשון |
| Since a root of a square is a thing, so we get a thing equals a number. | מפני כי שרש הצינסו יבא להיות הדבר א"כ היו לנו דבר שוה אל מספר |
|
|
דהיינו כל כך דברים כמו שהוא שרש אלף תר"כ מספרי' שוים אל מ' מספרי' |
|
|
שיבא לחלק מ' בשרש אלף תר"כ שיבא מזה באופן החלוק בשרשים שרש פ' חלקים מפ"א וככה יבא לשוות הדבר |
| Solved according to the said chapter. | ונעשה בעד הפרק האמור |
Chapter 21 |
פרק כ"א |
| When the cubes are equal to a root of a number:
|
כאשר המעוקבים יהיו שוים אל שרש מספר |
| The number of the cubes should be multiply by itself. | צריך להכות כמות המעוקבים בעצמם |
| Then, the numbers that are the radicand should be divided by that product. | ולחלק המספרים הנקובים שרש באותה ההכאה |
| Extract the square root of the cube root of the result, or vice versa the cube root of the square root, and this is the thing.
|
ומהעולה תקח השרש מרובע משרשו המעוקב או בהפך שרשו המעוקב משרשו המרובע וככה יבא לשוות הדבר |
|
עשה לי זה החשבון תמצא לי שני מספרים שיהיה חלק האחד מהאחר כמו מה שהוא ג' מד' וכשיוכה הראשון בעצמו ואותה ההכאה תכה בשני יעשה שרש כ' ורביע |
|
תעשה כמו שאומ' הכלל שלו |
|
תניח שהמספר הראשון היה ג' דברי' והשני ד' דברי' |
|
עתה תכה הראשון בעצמו שהוא ג' דברים ועולה ט' צינסי |
|
עתה תכה זאת ההכאה שהיא ט' צינסי במספר השני שהוא ד' דברים ועולה ל"ו מעוקבי' והם יבאו להיות שוים אל שרש כ' ורביע |
|
עתה תרדוף כפי הכלל הנתון |
|
דהיינו תכה כמות המעוקבים בעצמם ועולה אלף ורצ"ו |
|
אח"כ תחלק כ' ורביע שהוא כמות המספרים אשר נקבו להיות להם שרש על אלף ורצ"ו ועולה חלק אחד מס"ד |
|
ושרש מעוקב משרש מרובע מא' חלק מס"ד יהיה שוה הדבר |
|
ואתה הנחת שהמספר הראשון היה ג' דברי' א"כ תכה ג' בשרש מעוקב משרש מרובע מחלק א' מס"ד ועולה שרש מעוקב משרש מרובע או שרש מרובע משרש מעוקב מתשכ"ט מס"ד וזה יבא להיות א' וחצי וככה הוא המספר הראשון |
|
והמספר השני הונח היותו ד' דברים ולכן תכה ד' בשרש מעוקב משרש מרובע מא' חלק מס"ד ועולה שרש מעוקב משרש מרובע או אמור שרש מרובע משרש מעוקב מתצ"ו מס"ד אשר יבא להיות ב' וככה יבא להיות המספר השני |
| Know that this equation has the nature that fits chapter seven, although it exceeds by a square root. | ודע כי זאת ההשואה יש לו הטבע הנאות אל הפרק השביעי אע"פ שהולך יותר שרש אחד מרובע |
Chapter 22 |
פרק כ"ב |
| When numbers are equal to a root of cubes:
|
עוד כאשר המספרי' יהיו שוים אל שרשי המעוקבים |
| The numbers should be multiplied by themselves | צריך להכות המספרים בעצמם |
| Then, this product should be divided by the number of the cubes that are the radicand. | ולחלק ההכאה ההיא בכמות המעוקבי' הנקובים היות להם שרשים |
| The cube root of the result is equal to the thing.
|
ושרש המעוקב ממה שיעלה יבא לשוות הדבר |
|
תמצא לי שני מספרי' שיהיה חלק האחד מהאחר כמו שהוא ג' מה' וכשיוכה כל אחד על שרשו ויחוברו שתי אלו ההכאות יחד וזה הסך יוכה בשרש ח' יעשה מאה |
|
זהו כללו |
|
תניח שהמספר הראשון יהיה ג' דברים והאחר ה' דברים |
|
דע כי מן הדין היה שבעבור כי שרש הדבר אינו נכר שהיה ראוי שיונח המספר הראשון ג' צינסי והאחר ה' צינסי מפני שהצינסו יש לו היטב שרש שהוא דבר אחד |
|
וכמו זה ישוה מפני כי ככה ישוה ג' דברים מוכים בשרש ג' דברים כמו הכאת ג' צינסו בשרשו אשר יהיה כל כך דברים כמו שהוא שרש ג' |
|
עתה תכה המספר ראשון שהוא ג' דברים בשרשם שהוא שרש ג' דברים ועולה שרש כ"ז מעוקבים |
|
עתה תכה המספר השני שהוא ה' דברים בשרשם ועולה שרש קכ"ה מעוקבי' |
|
שרש כ"ז מעוקבי' ושרש קכ"ה מעוקבי' וזה הסך תכה בשרש ח' ויעלה שרש רי"ו מעוקבים ושרש אלף מעוקבים ואלו שני השרשים הם שוים אל ק' |
|
תרדוף כמו הכלל האמור למעלה |
|
תכה המספרי' שהם ק' ועולה עשרת אלפים |
|
ואלו תחלק על כמות המעוקבים הנקובים להיות להם שרש דהיינו בשרש רי"ו ובשרש אלף |
|
אשר זה תחלק באופן זה כפי התלמדות החלוק בשרשים תכה שרש אלף ושרש תשפ"ד בשרש אלף פחות שרש רי"ו אשר עולה תשפ"ד אשר אניח להיות מחלק |
|
ועתה אמור כן אם מתשפ"ד יבא שרש אלף פחות שרש רי"ו כמה יבא מאלף שהוא המספר שהוכה בעצמו |
|
תכה שרש אלף פחות שרש רי"ו באלף שעולה שרש מ 100000000 פחות שרש מ216000000 |
|
וזאת ההכאה תחלק בתשפ"ד אשר יעלה מזה שרש אלף תרכ"ו וכך חלקים 569344 מ614656 פחות שרש משנ"א וכך חלקים 255744 מ614656 |
|
ושרש מעוקב מזה שעלה יבא לשוות יבא לשוות הדבר |
|
| |
|
ואתה הנחת שהמספר הראשון יהיה ג' דברי' א"כ תכה ג' בשרש מעוקב מזה |
|
שצריך להשיב ג' אל שרש מרובע ואותו הרבוע צריך להשיב אל שרש מעוקב ויהיה לך שרש משרש מעוקב מתשכ"ט |
|
עתה תכה שרש משרש מעוקב מתשכ"ט בשרש משרש מעוקב מאלף תרכ"ו וחלקי' ה' מאות וס"ט אלפים ושמ"ד מתרי"ד אלפים ותרנ"ו פחות שרש משרש מעוקב משנ"א וחלקים רנ"ה אלפים ותשמ"ד מתרי"ד אלפים ותרנ"ו ועולה שרש משרש מעוקב מאלף אלפים וקפ"ו אלפים וכ"ט וחלקי' קנ"ח אלפים ותתקע"ו מתרי"ד אלפים ותרנ"ו פחות שרש משרש מעוקב מרנ"ו אלפים מקפ"ב וחלקי' קצ"ו אלפים ותר"ח מתרי"ד אלפים ותרנ"ו וככה יבא להיות המספר הראשון |
|
| |
|
ואתה הנחת שהמספר השני היותו ה' דברי' א"כ תכה ה' במה ששוה הדבר |
|
תשיב ה' אל שרש משרש מעוקב ויהיה לך שרש משרש מעו' מט"ו אלפים ותרכ"ה |
|
עתה תכה שרש משרש מעו' מט"ו אלפים ותרכ"ה בשרש משרש מעו' מאלף ותרכ"ו וחלקי' מתקס"ט אלפים ושמ"ד מתרי"ד אלפים ותרנ"ו פחות שרש משרש מעו' משנ"א וחלקים רנ"ה אלפים ותשמ"ד מתרי"ד אלפים ותרנ"ו ויעלה שרש משרש מעו' מכ"ה אלפי אלפים ות"כ אלפי' ותשכ"ג וחלקי' פ"ג אלפי' ותשי"ב מתרי"ד אלפי' ותרנ"ו פחות שרש מעו' משרש מה' אלפי אלפים ות"צ אלפים ותתע"ו וחלקי' קכ"א אלפים ושמ"ד מן תרי"ד אלפים ותרנ"ו |
|
| |
| Know that this equation is of the nature of chapter 7, even though it yields an extra root, since these two roots, i.e. the products received above, cannot be combined together to one root alone by their expression or their sum. | ודע כי זאת ההשואה היא מטבע הפרק השביעי אע"פ שתענה שרש אחד יותר מפני כי אותם שני שרשי' דהיינו ההכאות שנעשו למעלה אי אפשר לחברם יחד בענותם או בחברם בשרש אחד לבד |
Chapter 23 |
פרק כ"ג |
| When the squares of squares are equal to a root of a number:
|
כאשר הצינסי מצינסי יהיו שוים אל שרשי מספרי' |
| The number of the squares of squares should be multiplied by itself. | צריך להכות כמות הצינסי מצינסי בעצמם |
| Then, the number that is the radicand sould be divided by this product. | ולחלוק המספרי' הנקובי' על זאת ההכאה |
| Extract the root of the root of the root of the result and this is equal to the thing.
|
ותקח מהעולה שרש השרש מהשרש וככה יבא לשוות הדבר |
|
עשה לי זה החשבון תמצא לי מספר אחד שכאשר הוכה בשני שלישיו ואותה ההכאה תוכה בעצמה תעשה שרש חמשים אשאל כמה יבא להיות המספר האמור |
|
זהו כללו |
|
תניח שהמספר יהיה דבר אחד |
|
ויוכה דבר אחד בשני שלישיו ועולה ב' שלישי' מצינסו |
|
עתה תכה ב' שלישי' מצינסו בעצמו ועולה ד' תשיעיות מצינסו מצינסו שהם שוים אל שרש נ' |
|
עתה תרדוף כפי הכלל האמור למעלה |
|
תכה כמות הצינסי מצינסי בעצמם שהם ד' תשיעיות ויעלה י"ו חלקים מפ"א |
|
ותחלק המספרי' הנקובי' היות להם שרש ויעלה מזה רנ"ג ושמינית |
|
ושרש השרש משרשו יבא לשוות הדבר וככה יבא להיות המספר האמור |
| Know that this is almost the same as the nature of chapter 11, except that here a root of one degree higher is extracted. | ודע כי זה הוא כמעט דומה לטבע הפרק הי"א מלבד שלוקח שרש אחד יותר |
Chapter 24 |
פרק כ"ד |
| When the numbers are equal to a root of the squares of squares:
|
כאשר המספרים הם שוים אל שרשי הסינסי מצינסי |
| The numbers should be multiplied by themselves. | צריך להכות המספרי' בעצמם |
| Then, this product should be divided by the number of the squares of squares that are the radicand. | ולחלק אותה ההכאה בכמות הצינסי מצינסי הנקובי' היות להם שרש |
| The root of the root of the result should be extracted and this is equal to the thing.
|
ולקחת מהעולה שרש שרשו וככה יבא לשוות הדבר |
|
תמצא לי שני מספרי' שיהיה חלק האחד מהאחר כמו שב' הוא מג' וכשיוכה האחד באחר ואותה ההכאה תוכה בשרש ח' ועושה ק' |
|
זהו כללו |
|
תניח שהמספר ראשון ב' דברים והאחר מחוייב שיהיה ג' דברים |
|
עתה תכה הראשון בשני שהוא ב' דברי' על ג' דברים ועולה ו' צינסי |
|
תכה ו' צינסי על שרש ח' |
|
דע כי הנך צריך להשיב ו' צינסי אל שרשים והם בשהושבו אל שרשים יהיו שרש מל"ו צינסי מצינסי |
|
עתה תכה שרש מל"ו צינסי מצינסי בשרש ח' עולה שרש מרפ"ח צינסי מצינסי שהם שוים אל ק' מספרי' |
|
עתה תרדוף כפי הכלל האמור למעלה |
|
תכה המספרי' בעצמם שהם ק' ועולה עשרת אלפים |
|
ואלו תחלק על כמות הצינסי מצינסי הנקובים היות להם שרשים והם רפ"ח שעולה מזה ל"ד וי"ג חלקי' מי"ח וככה יבא לשוות הדבר |
|
ואתה הנחת שהמספר ראשון היה ב' דברי' א"כ תכה ב' על מה ששוה הדבר |
|
תשיב ב' אל שרש משרש שיבא להיות י"ו |
|
עתה תכה שרש משרש מי"ו בשרש שרש ל"ד וי"ג חלקים מי"ח ועולה שרש שרש מתקנ"ה וככה הוא המספר הראשון |
|
והשני הנחת היותו ג' דברים א"כ תכה שרש משרש ל"ד וי"ג חלקים מי"ח ועולה שרש משרש מאלפים ותתי"ב וחצי וככה יבא להיות המספר השני |
| Know that this equation can be returned to chapter 11. | ודע כי זאת ההשואה אפש' להשיבה אל הפרק הי"א |
| If the number of the squares of squares that are the radicand has a root, it can be returned to the second chapter. | ואם לכמות הצינסי מהצינס' הנקובים להיות להם שרש היה להם שרש היתה מושבת אל הפרק השני |
| Because its root is a square.
|
מפני כי שרשו היה צינסו |
| So, it becomes equal to the numbers.
|
ויבא להיות שוה אל המספרי' |
Chapter 25 |
פרק כ"ה |
| When things are equal to a root of things:
|
כאשר הדברים הם שוים אל שרשי הדברים |
| The [number] of the things should be multiplied by itself and this product is the divisor. | צריך להכות שרשי הדברים בעצמם ואותה ההכאה תחזיק למחלק |
| Divide [the number of] the things that are the radicand by the said product, i.e. by the square of the number of the things; the result is equal to the thing.
|
והדברי' אשר נקבו תחלק בהכאה האמורה דהיינו ברבוע כמות הדברי' ומה שיעלה ככה מספרי' יבא לשוות הדבר |
|
תמצא לי שני מספרי' שיהיה החלק האחד מהשני כמו שהוא ג' מד' וכן יעשה הראשון מוכה בח' כמו השני דהיינו שרשו מוכה בו' |
|
זהו כללו |
|
תניח שהראשון יהיה ג' דברי' והאחר ד' דברים |
|
עתה תכה הראשון שהוא ג' דברי' בח' ועולה כ"ד דברי' |
|
אח"כ תכה שרש השני שהוא שרש ד' דברים בו' ועולה שרש קמ"ד צינסי והוא שוה אל כ"ד דברי' |
|
עתה תרדוף כפי הכלל האמור למעלה |
|
תכה כמות הדברי' בעצמם שהוא כ"ד ועולה תקע"ו |
|
ותחלק הדברי' הנקובי' היות להם שרשי' והם קמ"ד על תקע"ו ועולה מזה א' רביע וזה יבא לשוות הדבר |
|
ואתה הנחת המספר ראשון היותו ג' דברים א"כ תכה ג' בא' רביע האמור ועולה ג' רביעי' ממספר דהיינו הג' דברי' אשר הנחת אותם בעד המספר הראשון וככה יבא להיות המספר הראשון |
|
ואתה הנחת היות המספר השני ד' דברים א"כ תכה הרביע האמור בד' ועולה ד' רביעים ממספר והם אחד שלם וככה יבא להיות המספר השני |
| Know that this equation can be returned to the third chapter: | ודע כי זאת ההשואה אפש' להשיבה אל הפרק השלישי |
| Since a root of a thing multiplied by a root of a thing yields a thing.
|
מפני כי שרש דבר מוכה בשרש דבר עושה דבר |
| A thing multiplied by a thing yields a square.
|
ודבר מוכה בדבר עושה צינסו |
| Hence, by multiplying each part [of the equation] by itself: | א"כ בהכות כל אחד מהחלקים בעצמו |
| The root of the things becomes things.
|
שרשי הדברים יבאו דברים |
| The things become squares.
|
והדברים יבאו צינסו |
| Then, by dividing the whole equation by the things, the aforesaid calculation is returned to the first chapter. | ובחלק אחר זה כל ההשואה על הדברי' ישוב החשבון האמור למעלה אל הפרק הראשון |
Chapter 26 |
פרק כ"ו |
| When the squares are equal to a root of things:
|
עוד כאשר הצינסו הם שוים אל שרשי דברים |
| The number of the squares should be multiplied by itself. | צריך להכות כמות הצינסי בעצמם |
| Then, the number of the things that are the radicand should be divided by this product, or by the square of [the number of] the squares. | ולחלק כמות הדברי' הנקובי' להיות להם שרשים על אותה ההכאה או רבוע הצינסי |
| Its cube root is equal to the thing.
|
ושרשו המעוקב יבא לשוות הדבר |
|
תמצא לי שני מספרי' שיהיה האחד חלק מהאחר כמו שב' הוא מה' וכשהוכה האחד בשני יעשה כמו הכאת שרש השני בח' |
|
זהו כללו |
|
תניח שהמספר הראשון יהיה ב' דברי' והאחר יהיה ה' דברי' |
|
עתה תכה הראשון בשני שהוא ב' דברי' על ה' דברי' ועולה עשרה צינסי ושמרם בעד חלק אחד מההשואה |
|
עתה תכה שרש השני שהוא שרש ה' בח' ועולה שרש מש"כ דברי' שהם שוים אל עשרה צינסי |
|
עתה תרדוף כפי הכלל האמור למעלה |
|
תכה כמות הצינסי בעצמם שהם עשרה ויעלה ק' |
|
עתה תחלק כמות הדברי' אשר נקבו היות להם שרשים והם ש"כ על ק' ויעלה מזה ג' וחומש |
|
ושרש המעוקב מג' וא' חומש יבא להיות הדבר |
|
ואתה הנחת שהמספר הראשון היה ב' דברים א"כ תכה ב' על שרש מעוקב מג' וא' חומש ויעלה שרש מעו' מכ"ה וג' חומשי' וככה יבא להיות המספר ראשון |
|
והשני הנחת היותו ה' דברים א"כ תכה ה' בשרש מעו' מג' וא' חומש ויעלה שרש מעו' מת' וככה יבא להיות המספר השני |
| Know that this equation can be returned to chapter 12, when each side [of the equation] is multiplied by itself. | ודע כי זאת ההשואה אפש' להשיבה אל הפרק הי"ב כאשר יוכה כל אחד מהחלקי' בעצמו |
| The result is things equal squares of squares.
|
והיה בא דברי' שוים אל צינסי מצינסי |
| After it is returned to the chapter 12, it can be returned to the seventh chapter, by dividing each side [of the equation] by a thing. | ועוד אפש' להשיבה אל הפרק השביעי אחר שהושבה אל הי"ב בחלק כל אחד מהחלקי' על דבר |
Chapter 27 |
פרק כ"ז |
| When the things are equal to a root of cubes:
|
כאשר הדברים יהיו שוים אל שרשי מעוקבים |
| The number of the things should be multiplied by itself. | צריך להכות כמות הדברים בעצמם |
| Then, this product should be divided by the number of the cubes that are the radicand and the result is equal to the thing.
|
ולחלק ההכאה ההיא על כמות המעוקבים הנקובי' היות להם שרש והעולה מזה ככה שוה הדבר |
|
תמצא לי שני מספרים שיהיה הראשון חלק מהשני כמו שב' הוא מג' וכשיוכה הראשון בשרש עצמו יעשה כמו הכאת השני בשנים |
|
זהו כללו |
|
תניח השני שהמספר הראשון יהיה ב' דברי' והשני ג' דברי' |
|
עתה תכה הראשון שהוא ב' דברים בשרש עצמו בזה האופן |
|
תשיב ב' דברי' אל שרש מרובע ויהיה לך שרש מד' צינסי |
|
אשר תכם בשרש ב' דברים דהיינו בשרש המספר הראשון ויעלה שרש ח' מעוקבים ושמרם בעד חלק מההשואה |
|
עתה תכה המספר האחר שהוא ג' דברים בב' ועולה ו' דברים שהם החלק האחר |
|
ויהיה לך שרש ח' מעוק' שוה לו' דברים |
|
עתה תרדוף כפי הכלל האמור למעלה |
|
תכה כמות הדברי' בעצמם והם ו' ועולה ל"ו |
|
ואלו הל"ו תחלק על כמות המעוקבי' הנקובי' להיות להם שרשים דהיינו על ח' ויבא ד' וחצי וככה שוה הדבר |
|
ואתה הנחת היות המספר הראשון ב' דברי' א"כ תכה ב' דברי' בד' וחצי ועולה ט' וככה שוה המספר הראשון |
|
והשני הנחת היותו ג' דברי' א"כ תכה ג' בד' וחצי ועולה י"ג וחצי וככה הוא המספר השני |
| Know that this equation can be returned to the ninth chapter, by multiplying each side of the equation by itself. | ודע שזאת ההשואה אפש' להשיבו אל הפרק התשיעי בהכות כל אחד מהחלקי' מההשואות בעצמו |
| After it is returned to the ninth chapter, it can then be returned to the first chapter by dividing each side [of the equation] by a square. | ובהיותו מושב אל הפרק התשיעי אפשר להשיבה אל הפרק הראשון בחלק כל חלק על צינסי |
| By dividing the said equation by a thing, it is returned to the third chapter in the aforesaid way. | ובחלק ההשואה האמורה על דברים תשוב אל הפרק השלישי באופן האמור |
Chapter 28 |
פרק כ"ח |
| עוד באופן אחר | |
| When the things are equal to a root of squares of squares:
|
כאשר הדברים יהיו שוים אל שרשי צינסו מצינסי |
| The number of the things should be multiplied by itself. | צריך להכות כמות הדברים בעצמם |
| Then, this product should be divided by the number of the squares of squares that are the radicand. | ולחלק אותה ההכאה על כמות הצינסי מצינסי הנקובי' היות להם שרש |
| The root of the result is equal to the thing.
|
ושרש העולה יבא להיות שוה הדבר |
|
עשה לי זה החשבון תמצא לי ב' מספרי' שיהיה האחד חלק מהאחר כמו שהוא ג' מה' וכשיוכה האחד באחר ואותה ההכאה תוכה בשרש ח' יעשה כמו המספר השני |
|
זהו כללו |
|
תניח שהמספר ראשון יהיה ג' דברים והשני יהיה ה' דברים |
|
עתה תכה האחד באחר דהיינו ג' דברים בה' דברי' עולה ט"ו צינסי |
|
עתה תכה זאת ההכאה שהיא ט"ו צינסי בשרש ח' |
|
וזכור כי הנך צריך להשיב הט"ו צינסי אל שרש ויהיה לך שרש מרכ"ה צינסי מצינסי |
|
ותכם בשרש ח' ויהיה לך שרש מאלף ת"ת צינסי מצינסי ושמרם בעד חלק אחד מההשואה |
|
א"כ יהיה לך ה' דברי' שוים אל שרש אלף ת"ת צינסי מצינסי |
|
עתה תרדוף כפי הכלל האמור למעלה |
|
תכה כמות הדברים בעצמם והם ה' ועולה כ"ה |
|
וזאת ההכאה רצוני כ"ה תחלק בכמות הצינסי מצינסי הנקובים להיות להם שרש שהם אלף ת"ת ויבא מזה חלק אחד מע"ב |
|
ושרש זה החלק מע"ב יבא להיות הדבר |
|
ואתה הנחת היות המספר הראשון ג' דברים א"כ תכה ג' בשרש חלק א' מע"ב ועולה שרש מט' חלקים מע"ב שיבא להיות שרש מא' חלק מח' וככה יבא להיות המספר ראשון |
|
והשני הנחת היותו ה' דברי' א"כ תכה ה' בשרש א' חלק מע"ב ועולה שרש מכ"ה חלקים מע"ב וככה יבא להיות המספר השני |
| Know that this equation can be returned to the second chapter, and also can be returned to chapter 13: | ודע כי זאת ההשואה אפש' להשיבה אל הפרק השני וג"כ אפש' להשיבה אל הפרק הי"ג |
| First to chapter 13: by multiplying each side [of the equation] by itself. | [46]וראשונה אל הי"ג בהכות כל אחד מהחלקי' בעצמו |
| The things become squares.
|
הדברי' יבאו צינסי |
| The root of squares of squares becomes squares of squares.
|
ושרש צינסו מצינסו יבא צינסו מצינסו |
| By dividing also each side by a square: | ובחלוק ג"כ כל חלק על צינסו |
| The squares become numbers. | הצינסי יבאו אל מספרי' |
| The squares of squares become squares. | והצינסי מצינסי יבאו צינסי |
| Thus, it is returned to the second chapter. | והיה מושב אל הפרק השני |
| If you wish it can be returned to the third chapter: | וברצותך להשיבה אל הפרק השלישי |
|
יהיו לך ה' דברי' שוים אל כך צינסי כמו שהוא שרש אלף ת"ת ויבא לך לחלק ה' על שרש אלף ת"ת ומה שיעלה שוה הדבר |
Chapter 29 |
פרק כ"ט |
| When the squares are equal to a root of squares:
|
כאשר הצינסי יהיו שוים אל שרשי צינסי |
| The number of the squares should be multiplied by itself. | צריך להכות כמות הצינסי בעצמם |
| Then, [the number of] the squares that are the radicand should be divided by this product. | ולחלוק הצינסי הנקובי' להיות להם שרש על אותה ההכאה |
| The root of the result is equal to the thing.
|
ושרשרש העולה יבא לשוות הדבר |
|
תמצא לי ב' מספרי' שיהיה הראשון חלק מהשני כמו שד' הוא מה' ומוכה האחד באחר יעשה כמו הכאת השני בשרש ח' |
|
זהו כללו |
|
תניח שהמספר ראשון ד' דברי' והשני יהיה ה' דברי' |
|
עתה תכה הראשו' בשני דהיינו ד' דברי' בה' דברי' ועולה כ' צינסי ושמרם בעד חלק אחד מן חלקי ההשואה |
|
תכה המספר השני שהוא ה' דברי' בשרש ח' |
|
וזכור כי הנך צריך להשיב הדברי' אל שרשי' ויהיה לך שרש כ"ה צינסי |
|
ותכם בשרש ח' ועולה שרש מר' צינסי שהם שוים אל כ' צינסי |
|
עתה תרדוף כפי הכלל האמור למעלה |
|
תכה כמות הצינסי בעצמם והם כ' ועולה ת' |
|
עתה תחלק כמות הצינסי אשר נקבו להיות להם שרשי' שהם ר' על אלו הת' ויבא חצי |
|
ושרש החצי יבא להיות הדבר |
|
ואתה הנחת היות המספר ראשון ד' דברי' א"כ תכה ד' בשרש חצי יעלה שרש ח' וככה יבא לשוות המספר ראשון |
|
והשני הנחת ה' דברים א"כ תכה ה' בשרש חצי ועולה שרש מי"ב וחצי וככה יבא להיות המספר השני |
| Know that this equation can be returned to chapter 13, by multiplying each side [of the equation] by itself, then dividing it by roots; it is converted to squares of squares equal squares.
|
ודע כי זאת ההשואה אפשר להשיבה אל הפרק הי"ג בהכות כל חלק בעצמו ולחלקו בשרשי' ויבאו צינסי מצינסי שוים אל צינסי |
| Or, if you wish, divide again by things; it is returned to the eighth chapter. | ואם רצית לחלק אח"כ על דברי' תשוב אל הפרק השמיני |
Or, if you wish, divide it by squares; it is returned to the second chapter, i.e. numbers equal squares.
|
וברצותך לחלקה על צינסי תשוב אל הפרק השני דהיינו מספרי' שוים אל צינסי |
Chapter 30 |
פרק שלשים |
| When the squares are equal to a root of cubes:
|
כאשר הצינסי יהיו שוים אל שרשי המעוקבי' |
| The number of the squares should be multiplied by itself. | צריך להכות כמות הצינסי בעצמם |
| Then, the number of the cubes that are the radicand should be divided by this product; the result is equal to the thing.
|
ולחלק כמות המעוקבי' הנקובי' להיות להם שרש על אותה ההכאה והעולה מזה ככה ישוה הדבר |
|
תמצא לי ב' מספרי' שיהיה האחד חלק מהאחר כמו ב' מג' ובהכות הראשו' בשרשו יעשה כמו הכאת השני בעצמו |
|
וזהו כללו |
|
תניח שהמספר ראשון היה ב' דברי' והאחר ג' דברי' |
|
תכה הראשון שהוא ב' דברי' בשרשם |
|
זכור לך כי אתה צריך להשיב ב' דברי' אל שרשי' ויהיה לך שרש מד' צינסי |
|
אשר אתה צריך להכות [47]בשרש ב' דברי' ועולה שרש ח' מעוקב ושמר זה בעד חלק אחד מההשואה |
|
עתה תכה המספר האחר שהוא ג' דברי' בעצמו ועולה ט' צינסי שהם שוים אל שרש מעוקב ח' |
|
עתה תרדוף כפי הכלל הנתון למעלה |
|
תכה כמות הצינסי בעצמם שהם ט' ועולה פ"א |
|
עתה תחלק כמות המעוקבי' הנקובי' להיות להם שרש שהם ח' על אלו הפ"א ויבא מזה ח' חלקי' מפ"א וככה שוה הדבר |
|
ואתה הנחת היות המספר ראשון ב' דברי' א"כ תכה ב' בח' חלקי' מפ"א ועולה י"ו חלקי' מפ"א וככה הוא המספר ראשון |
|
והשני הנחת היותו ג' דברי' א"כ תכה ג' בח' חלקי' מפ"א ועולה כ"ד חלקי' מפ"א וככה הוא המספר השני |
| Know that this equation can be returned to the tenth chapter, by dividing by roots: | ודע כי זאת ההשואה אפשר להשיבו אל הפרק העשירי בחלוק על שרשים |
|
מפני כי ט' צינסי הם שרש מפ"א צינסי אשר הם שוים אל ח' שרשי' מעו' |
|
א"כ בהכאת ט' צינסי בעצמם אשר הם שרש מפ"א צינסי מצינסי עושים פ"א צינסי מצינסי |
|
ולהכות שרש ח' מעוקבי' בעצמו עושה ח' מעוקבי' |
|
א"כ יהיה לך פ"א צינסי מצינסי שוים אל ח' מעוקבי' וזהו האופן לחלק בשרשי' |
| When we wish to divide the equation by a thing, it brings you to the ninth chapter. | וברצותנו לחלק זאת ההשואה על דברי' תביאך אל הפרק התשיעי |
| By dividing it by a square, it brings you to the third chapter. | ובחלוק אותה על צינסי תביאך אל הפרק השלישי |
| If you divide it by a cube, it brings you to chapter 1. | ואם תחלקנה על מעו' תביאך אל פרק א' |
Chapter 31 |
פרק ל"א |
| עוד רצוני להראותך באופן אחר | |
| When the cubes are equal to a root of squares:
|
כאשר המעוקבי' יהיו שוים אל שרשי צינסי |
| The number of the cubes should be multiplied by itself. | צריך להכות כמות המעוקבי' בעצמם |
| Then, the number of the squares that are the radicand should be divided by this product. | ולחלק כמות הצינסי הנקובי' להיות להם שרש על אותה ההכאה |
| Extract the root of the root of the quotient and this is the thing.
|
ותקח שרש השרש מהעולה בחלוק וככה ישוה הדבר |
|
תמצא לי מספר אחד שכשיוכה בשני שלישיו ואותה ההכאה תוכה במספר האמור יעשה כמו הכאת המספר ההוא בשרש ח' אשאל כמה הוא כל אחד מהמספרי' |
|
זהו כללו |
|
תניח שהמספר הוא דבר אחד |
|
תכה עתה דבר אחד בב' שלישיו ועולה ב' שלישי צינסו |
|
וזאת ההכאה תכה במספר האמור דהיינו בדבר אחד ועולה ב' שלישי מעוקב ושמור זה בעד חלק אחד מההשואה |
|
אח"כ תכה המספר האמור רצוני דבר אחד בשרש ח' |
|
ותזכור להשיב הדברי' אל שרש ויהיה לך שרש מא' צינסו |
|
אשר תכהו בשרש ח' ועולה שרש ח' צינסי שהם שוים אל ב' שלישי מעוקב |
|
עתה תרדוף כפי הכלל האמור למעלה |
|
תכה כמות המעוקבי' בעצמו והוא ב' שלישי' ועולה ד' תשיעיות |
|
ותחלק כמות הצינסי הנקובי' להיות להם שרש והם ח' על אלו הד' תשיעיו' ויבא מזה י"ח |
|
ושרש השרש מי"ח יבא להיות הדבר וכן הוא המספר האמור |
| Know that this equation can be returned to chapter 11, by dividing by roots: | ודע כי זאת ההשואה אפשר להשיבה אל הפרק הי"א בזה האופן בחלק בשרשי' |
| As you mentioned roots in one side, convert the other side into roots: so, multiply the cubes by themselves; you get a root of squares that is equal to a root of squares of squares [of squares].
|
כי כמו שנקבת שרשי' בחלק בצד האחד כן ג"כ תשיב החלק הצד האחר אל שרשים תכה א"כ המעוקבי' בעצמם ויהיה לך שרשי צינסי שוים אל שרשי צינסי מצינסי |
| Since cubes by cubes generate cubes of cubes that are squares of squares of squares.
|
[48]מפני כי מעו' במעו' עושה מעו' המעו' אשר הם באי' להיות צינסי דצינסי דצינסי |
| So, by dividing by a root the result is squares equal squares of squares of squares.
|
א"כ בחלוק בשרשי' יבא צינסו שוה אל צינסו דצינסו מצינסו |
| By dividing by a square the result is a number that is equal to squares of squares.
|
אשר זה בחלוק בצינסי יבא ממנו מספר שוה לצינסו מצינסו |
| In this way that you have seen it can be returned properly. | ובאופן הזה אשר ראית אפשר להשיבה מאד היטב |
Chapter 32 |
פרק ל"ב |
| עשה לי זה החשבון אשר אומר אליך פה בקרוב | |
| אבל קודם זה רצוני להבינך טבע זה הפרק וזה הוא | |
| When squares of squares are equal to a root of squares:
|
כאשר צינסי מצינסי יהיו שוים אל שרשי' מצינסי |
| The number of the squares of squares should be multiplied by itself. | צריך להכות כמות הצינסי מצינסי בעצמם |
| Then, the number of the squares that are the radicand should be divided by this product. | ולחלק כמות הצינסי הנקובי' להיות להם שרש על אותה הכאה |
| The square root of the cube root of the result is equal to the thing.
|
ושרש המרובע משרש המעו' ממה שיעלה יבא לשוות הדבר |
|
תמצא לי שני מספרים שיהיה הראשון חלק מהשני כמו שהוא ב' מג' ויוכה הקטון בגדול ואותה ההכאה תוכה בעצמה יעשה כמו הכאת השני בשרש ח' |
|
זהו כללו |
|
תשים שהמספר ראשון יהיה ב' דברי' |
|
תכה הקטון בגדול וזהו ב' דברי' בג' דברי' ועולה ו' צינסי |
|
אח"כ תכה זאת ההכאה שהיא ו' צינסי ועולה ל"ו צינסי מצינסי ושמור זה בעד אחד מההשואה |
|
אח"כ תכה השני שהוא הגדול שהוא ג' דברי' בשרש ח' |
|
וזכור כי אתה צריך להשיב ג' דברי' אל שרש ויהיה לך שרש מט' צינסי |
|
ותכהו על שרש ח' ועולה שרש מע"ב צינסי שהם שוים אל ג' צינסי מצינסי |
|
עתה תרדוף כפי הכלל האמור למעלה |
|
תכה כמות הצינסי מצינסי בעצמם שזהו ל"ו בעצמם ועולה אלף ורצ"ו |
|
וחלק כמות הצינסי הנקובי' להיות להם שרש שהם ע"ב על אלו אלף ורצ"ו שיבא ממנו חלק אחד מי"ח |
|
ושרש המרובע משרש המעו' או אמור השרש המעו' משרש המרובע מחלק אחד מי"ח יבא לשוות הדבר |
|
ואתה הנחת היות המספר הראשון ב' דברי' א"כ תכה ב' בשרש מרובע משרש מעו' מחלק א' מי"ח ועולה שרש מעו' משרש מרובע מס"ד חלקי' מי"ח אשר הם שרש מרובע משרש מעו' מג' וה' תשיעיות וככה יבא להיות המספר ראשון |
|
והשני הנחת היותו ג' דברי' א"כ תכה ג' בשרש מעו' משרש מרובע מחלק א' מי"ח ועולה שרש מרובע משרש מעו' מתשכ"ט חלקי' מי"ח שזהו מ' וחצי וככה הוא המספר השני |
| Know that this equation can be returned to chapter 21. | ודע כי זאת ההשואה אפשר להשיבה אל הפרק הכ"א |
Chapter 33 |
פרק ל"ג |
| When the cubes are equal to a root of cubes:
|
כאשר המעוקבי' יהיו שוים אל שרשי מעוקבי' |
| The number of the cubes should be multiplied by itself. | צריך להכות כמות המעוקבי' בעצמם |
| Then, the number of the cubes that are the radicand should be divided by this product. | ולחלק כמות המעוק' הנקובי' להיות להם שרש באותה ההכאה |
| The cube root of the result is equal to the thing.
|
ושרש המעו' מהעולה מזה יבא לשוות הדבר |
|
תמצא לי שני מספרי' שיהיה האחד חלק מהשני כמו שב' הוא מג' ומוכה הראשון בעצמו ואותה ההכאה תוכה במספר השני יעשה [49]כמו הכאת השני בשרשו |
|
זהו כללו |
|
תניח שהמספר ראשון יהיה ב' דברי' והאחר יבא להיות ג' דברים |
|
עתה תכה הראשון שהוא ב' דברים בעצמם ועולה ד' צינסי |
|
עתה תכה זאת ההכאה שהיא ד' צינסי במספר השני שהוא ג' דברי' ועולה י"ב מעוקבי' ושמרם בעד חלק אחד מההשואה |
|
אח"כ תכה המספר השני שהוא ג' דברים בשרש ג' דברי' |
|
ותזכור כי הנך צריך להשיב ג' דברי' אל שרש ויהיה לך שרש מט' צינסי |
|
וזה השרש מט' צינסי תכה בשרש ג' דברי' ועולה שרש מרע"ה מעוקבי' שהם שוים אל י"ב מעוקבי' |
|
עתה תרדוף כפי הכלל האמור למעלה |
|
תכה כמות המעו' בעצמם וזהו י"ב עולה קמ"ד |
|
ותחלק כמות המעוקבי' הנקובי' להיות להם שרש וזהו י"ב על קמ"ד ויבא מזה ג' חלקי' מי"ו |
|
ושרש מעו' מג' חלקי' מי"ו יבא לשוות הדבר |
|
ואתה הנחת היות המספר ראשון ב' דברי' א"כ תכה ב' בשרש מעו' מג' חלקי' מי"ו ועולה שרש מעו' |
|
והשני הנחת היותו ג' דברי' א"כ תכה ג' בשרש מעו' מג' חלקי' מי"ו ועולה שרש מעו' מה' חלקי' מי"ו וככה הוא המספר השני |
| Know that this equation can be returned to the seventh chapter, by multiplying each side [of the equation] by itself. | ודע כי זאת ההשואה אפשר להשיבה אל הפרק השביעי בהכות כל אחד מהחלקי' בעצמו |
| By dividing the product by a cube, the result is numbers equal cubes. | ויבא בחלוק ההכאה על מעוקבי' מספרי' שוים אל מעוקבי' |
| A root of cubes by a root of cubes generate cubes.
|
ועל שרשי מעוקבי' בשרשי מעו' עושה מעוקבי' |
| Cubes by themselves generate cubes of cubes.
|
ומעוקבי' בעצמם עושה מעו' ממעו' |
| Hence, by dividing cubes by cubes the result is numbers.
|
א"כ בחלוק מעו' על מעו' יעלה מהם מספרים |
| And cubes of cubes by cubes the result is cubes.
|
ומעוקבי' ממעוקבי' על מעוקבי' יבא מהם מעוקבי' |
| By dividing by squares, the result is things equal squares of squares, so it is returned to chapter 12. | ובחלוק על צינסי יבא מזה דבר שוה אל צינסו מצינסו ויבא להיותה מושבת אל הפרק הי"ב |
| We proceed according to the aforesaid chapter. | ונעשה עם הפרק האמור למעלה |
Chapter 34 |
פרק ל"ד |
| When the cubes are equal to a root of squares of squares:
|
כאשר המעו' הם שוים לשרשי צינסי מצינסי |
| The number of the cubes should be multiplied by itself. | צריך להכות כמות המעוקבי' בעצמם |
| Then, the number of squares of squares that are the radicand should be multiplied by this product. | ולחלק כמות הצינסי מצינסי הנקובי' היות להם שרש על אותה ההכאה |
| The root of the result is equal to the thing.
|
והעולה מזה הנה שרשו ישוה הדבר |
|
עשה לי זה החשבון תמצא לי שני מספרי' שיהיה האחד חלק מהאחר כמו שג' הם מה' ומוכה הראשון בעצמו ואותה ההכאה תוכה |
|
זהו כללו |
|
תניח שהמספר ראשון יהיה ג' דברי' והשני יהיה ה' דברי' |
|
עתה תכה הראשון בעצמו שהוא ג' דברי' ויעלה ט' צינסי |
|
ואלו |
|
אח"כ תכה המספר השני שהוא ה' דברי' בעצמו ועולה כ"ה צינסי |
|
אח"כ תכה זאת ההכאה רצוני כ"ה צינסי בשרש [50]ח' |
|
ותזכור כי הנך צריך להשיב כ"ה צינצי אל שרש ויהיה לך שרש מתרכ"ה צינסי מצינסי |
|
ותכם בשרש ח' ועולה שרש ה' אלפי' צינסי מצינסי שהם שוים אל מ"ה מעוקבי' |
|
עתה תרדוף כפי הכלל האמור למעלה |
|
תכה כמות המעוק' בעצמם וזהו מ"ה מעו' ועולה אלפיים וכ"ה |
|
ותחלק כמות הצינסי מצינסי הנקובי' להיות להם שרש והם ה' אלפים על אלו אלפיים וכ"ה ויבא מזה ב' שלמים ול"ח מפ"א |
|
ושרש אלו הב' ול"ח מפ"א יבא לשוות הדבר |
|
ואתה הנחת היות המספר הראשון ג' דברי' א"כ תכה ג' בשרש ב' ול"ח מפ"א ועולה שרש כ"ב וב' תשיעיות וככה יבא להיות המספר הראשון |
|
והשני הנחת היותו ה' דברי' א"כ תכה ה' בשרש ב' ול"ח מפ"א ועולה שרש מס"א ונ"ט מפ"א וככה יבא להיות המספר השני |
| Know that if you wish to return it to one of the other chapters, multiply each side [of the equation] by itself; the squares of squares become equal to cubes of cubes, or squares of squares of squares.
|
ודע שאם רצית להשיבה אל פרק מה מהאחרי' תכה עתה כל אחד מן החלקי' בעצמו והיה עולה צינסי מצינסי אל מעוק' המעוק' או שוה אל צינסו מצינסו מצינסו |
| Divide this equation by a square; it becomes squares equal squares of squares and it is returned to chapter 13.
|
וזאת ההשואה בחלוק [אותה][51] על צינסי יעלה יבא צינסי שוה אל צינסו מצינסו והיתה מושבת אל הפרק הי"ג |
| Dividing it by a cube; it becomes things equal cubes and it is returned to chapter 8.
|
ובחלקה על מעו' יבא דבר שוה אל מעו' והיתה מושבת אל פרק ח' |
| Dividing it by a square of a square, it is returned to the second chapter, which is numbers equal squares.
|
ובחלקה על צינסי מצינסו היתה באה לך |
Chapter 35 |
פרק ל"ה |
| When the squares of squares are equal to [a root of] squares of squares:
|
עוד באופן אחר דהיינו כאשר הצינסי מצינסי הם שוים אל צינסי מצינסי |
| The number of squares of squares should be multiplied by itself. | צריך להכות כמות הצינסי מצינסי בעצמם |
| Then, the number of squares of squares that are the radicand should be divided by this product. | ולחלק כמות הצינסי מצינסי הנקובי' להיות להם שרש על ההכאה ההיא |
| The root of a root of the result is equal to the things.
|
ושרש שרש מהעולה יבא לשוות הדבר |
|
תמצא לי שני מספרי' שיהיה האחד חלק מהאחר כאשר ה' הוא מז' ומוכה הראשון בשני ואותה ההכאה תוכה בעצמה יעשה כמו הכאת המספר הקטן בעצמו וההכאה ההיא תוכה בשרש ח' |
|
זהו כללו |
|
תניח שהמספר הראשון יהיה ה' דברי' והאחר ז' דברי' |
|
עתה תכה הראשון בשני וזהו ה' דברי' בז' דברי' ועולה ל"ה צינסי |
|
אח"כ תכה זאת ההכאה רצוני ל"ה צינסי בעצמם ועולה אלף ורכ"ה צינסי מצינסי ושמור בעד אחד מחלקי ההשואה |
|
אח"כ תכה המספר הקטו' שהוא ה' דברי' בעצמו ועולה כ"ה צינסי |
|
ותכה אלו הכ"ה צינסי בשרש ח' |
|
וזכור כי הנך צריך להשיב כ"ה צינסי אל שרש ויהיה לך שרש מתרכ"ה צינסי מצינסי |
|
ותכם בשרש ח' ועולה שרש ה' אלפי' צינסי מצינסי שהם שוים אל אלף ורכ"ה צינסי מצינסי |
|
עתה תרדוף כפי הכלל האמור למעלה |
|
תכה כמות הצינסי בצינסי בעצמם והם אלף ורכ"ה ועולה אלף אלפי' ות"ק אלפי' ותרכ"ה |
|
ותחלק כמות הצינסי מצינסי הנקובי' להיות להם שרש בהשואה שהם ה' אלפי' על אלף אלפים ות"ק אלפי' ותרכ"ה ויבא מזה ח' חלקי' מאלפיים ות"א |
|
ותקח שרש השרש מאלו [52]הח' חלקי' מאלפיים ות"א וככה יבא לשוות הדבר |
|
ואתה הנחת היות המספר ראשון ה' דברי' א"כ תכה ה' בשרש שרש מח' חלקי' מאלפיים ת"א ועולה שרש משרש ב' וקצ"ח חלקי' מאלפיים ות"א וככה יבא להיות המספר ראשון |
|
והשני הנחת היותו ז' דברי' א"כ תכה ז' בשרש שרש מח' חלקי' מאלפיים ות"א ועולה שרש משרש ח' וככה יבא להיות המספר השני |
| Know that this calculation can be returned to chapter 11, by multiplying each side of the equation by itself: | ודע כי זה החשבון אפשר להשיבו אל הפרק הי"א בזה האופן בהכות כל אחד מחלקי ההשואה בעצמו |
| The root of squares of squares becomes squares of squares.
|
ושרש צינסו מצינסו יבא צינסו מצינסו |
| The squares of squares become squares of squares of squares of squares.
|
והצינסי מצינסי יבא צינסו מצינסו מצינסו מצינסו |
| Then, dividing it by squares of squares: | ואח"כ בחלוק זה על צינסו מצינסו |
| The squares of squares become numbers.
|
יבאו לך הצינסי מצינסי מספרי' |
| And the squares of squares of squares of squares become squares of squares.
|
והצינסי מצינסי מצינסי מצינסי יבאו צינסי מצינסי |
| So, it is returned to the said chapter 11, as we intended to return it. | והיה מושב אל הפרק הי"א האמור |
Chapter 36 |
פרק ל"ו |
| When things are equal to numbers and a root of numbers:
|
כאשר הדברי' יהיו שוים אל מספרי' ואל שרשי מספרי' |
| The numbers that are [not] the radicand should be divided by the number of the things, and the result is kept. | צריך לחלק כמות המספרי' הנקוב היות לו שרש על |
| Then, the number of the things should be multiplied by itself. | ואח"כ להכות כמות הדברי' בעצמם |
| The number that is the radicand should be divided by this product. | ולחלק המספרי' הנקובי' להיות להם שרש על אותה הכאה |
| Add the root of the result to the quotient we said should be kept; the result is equal to the thing and it is a number and a root.
|
ושרש העולה מזה תחבר אל מה שעלה מהחלוק שאמרנו לשמרו ומה שיעלה ישוה הדבר ויהיה מספר ושרש |
|
תמצא לי שני מספרי' שיהיה האחד מהאחר כמו שב' הוא מג' ומוכה הראשון בה' והשני בז' ושתי אלו ההכאות יחוברו יחד יעשו י"ו ושרש ח' |
|
זאת היא פעלת זה החשבון |
|
תניח שהמספר ראשון יהיה ב' דברי' והשני ג' דברי' |
|
עתה תכה הראשון שהוא ב' דברי' בה' ויעלה עשרה דברי' |
|
אח"כ תכה המספר השני שהוא ג' דברי' בז' ועולה כ"א דברי' |
|
וחברם עם ההכאה הראשונה שהיא י' דברי' ויהיה לך ל"א דברי' ושמרם בעד המנגד מהחלק האחד |
|
ויהיה לך ל"א דברי' שוים אל י"ו ושרש ח' |
|
עתה תרדוף כפי הכלל האמור למעלה |
|
תחלק המספרי' בכמות הדברי' וזהו י"ו על ל"א אשר יעלה מזה י"ו מל"א ושמור זה |
|
אח"כ תשיב כמות הדברי' אל שרש שהם ל"א ועולה תתקס"א |
|
ותחלק המספרי' הנקובי' להיות להם שרש שהם ח' על תתקס"א ועולה מזה ח' מתתקס"א וככה שוה הדבר |
|
ואתה הנחת היות המספר ראשון ב' דברי' א"כ תכה ב' בי"ו מל"א ושרש ח' מתתקס"א ועולה א' וא' מל"א ושרש ל"ב מתתקס"א וככה יבא להיות המספר ראשון |
|
והשני הנחת היותו ג' דברי' א"כ תכה ג' בי"ו מל"א ושרש ח' מתתקס"א ועולה א' וי"ז מל"א ושרש ע"ב מתתקס"א וככה הוא המספר השני |
| This calculation is done according the the rule of [the present] chapter, as well as] the first chapter and [chapter] 18. | ויבא להיות נעשה זה החשבון מן הכלל [הפרק][53] הראשון והי"ח |
Chapter 37 |
הפרק הל"ז |
| When numbers are equal to things and a root of things:
|
כאשר המספרי' הם שוים אל דברי' ושרשי דברי' |
| The numbers should be divided by the number of the things that are not the radicand. | צריך לחלק המספרי' בכמות הדברי' שאינם נקובי' להיות להם [54]שרש |
| Then, the number of the things that are [not] the radicand should be multiplied by itself. | ואח"כ להכות כמות הדברי' הנקובי' להיות להם שרש בעצמם |
| The number of the things that are the radicand should be divided by this product. | ולחלק כמות הדברי' הנקובי' להיות להם שרש על אותה ההכאה |
| Add a quarter of the result to the quotient of the numbers divided by the number of the things. | ורביע מהעולה תחבר על המספר הבא בחלוק המספר על כמות הדברי' |
| When the root of the quarter that is added to the number - i.e. the quotient of the number of the things that are the radicand divided by the product of the number of the things that are not the radicand - is subtracted from the root of the sum, the result is the root of the thing. | ושרש זה הסך בהיות מוצא שרש מהרביע אשר חובר אל המספר דהיינו מהחלוק הבא לך בחלוק כמות הדברי' הנקובי' להיות להם שרש על הכאת כמות הדברי' הבלתי נקובי' להיות להם שרש יבא להיות שרש הדבר |
| When it is multiplied by itself, the result is equal to the thing.
|
ובהכות זה על עצמו יבא לשוות הדבר |
|
והנה המשל תמצא לי שני מספרי' שיהיה האחד חלק מאחר כמו שב' הוא מג' ובהכות הראשון בג' ושרש השני בד' ויחוברו שתי אלו ההכאות יחד יעשה שלשים |
| The procedure: | זהו מעשהו |
|
תניח שהמספר ראשון יהיה ב' דברי' והאחר יהיה ג' דברים |
|
|
עתה תכה הראשון שהוא ב' דברי' בג' עולה ו' דברי' ושמרם |
|
|
אח"כ תכה שרש השני שזה הוא שרש מג' דברי' בד' |
|
ותזכור כי הנך צריך להשיב ד' אל שרש ויהיה לך שרש מי"ו |
| ותכה זה בשרש ג' דברי' ועולה שרש מ"ח דברי' | |
|
ותחבר שתי אלו ההכאות יחד |
|
|
וזהו ו' דברי' עם שרש מ"ח דברי' |
|
|
ויהיה לך ו' דברי' ושרש ממ"ח דברי' והם באים להיות שוים אל ל' |
|
עתה תרדוף כפי הכלל האמור למעלה |
| תחלק המספרי' בכמות הדברי' הנקובי' להיות להם שרש והם ל' על ו' ועולה מזה ה' ושמרהו | |
| אח"כ תכה כמות הדברי' אשר לא נוקבו להיות להם שרש שהם ו' בעצמם ועולה ל"ו | |
| ותחלק כמות הדברי' הנקובי' להיות להם שרש שהם מ"ח על ל"ו ויבא מזה א' ושליש | |
| ותחבר הרביע מא' ושליש עם המספר אשר שמרת והוא על ה' ויהיה לך ה' ורביע | |
| ושרש זה הסך פחות שרש א' רביע אשר בא לנו בחלוק כמות הדברי' אשר | |
| והוא מוצא שרש א' שליש מהשרש מחבור ה' וא' שליש וזהו ה' ושליש יבא להיות שרש הדבר האמור | |
| ואותו תכפול בעצמו באופן זה באמור שרש ה' וא' שליש פחות שרש מא' שליש מוכה בשרש ה' וא' שליש פחות שרש א' שליש שעולה בה' וב' שלישי פחות שרש מס"ד תשיעיות | |
| אשר זה השרש הוא ב' וב' שלישי | |
| ותוציאהו מה' וב' שלישי וישאר ג' וככה | |
|
| |
|
|
ואתה הנחת היות המספר ראשון ב' דברי' א"כ תכה ג' בב' עולה ו' וככה יבא להיות המספר ראשון |
|
|
ואתה הנחת שהשני היה ג' דברי' והדבר יבא להיות ג' א"כ תכה ג' בג' וככה יבא להיות המספר השני |
Chapter 38 |
פרק ל"ח |
| When squares are equal to numbers and a root of numbers:
|
כאשר הצינסי יהיו שוים אל מספרי' ולשרשי מספרי' |
| The numbers should be divided by the number of the squares. | צריך לחלק המספר על כמות הצינסי והעולה תשמור |
| Then, the number of the squares should be multiplied by itself. | ואח"כ להכות כמות הצינסי בעצמם |
| The numbers that are the radicand should be divided by this product. | ולחלק המספרי' אשר נקבו להיות להם שרש על אותה ההכאה |
| The root of the quotient is added to the number you kept, i.e. the result of division of the numbers by the number of the squares; the sum is equal to the square. | ושרש חלק מה שיעלה מחובר עם המספר אשר שמרת דהיינו מאותו שעלה לך בחלוק המספרי' על כמות הצינסי ומה שיעלה הסך ישוה הצינסו |
| The root of the mentioned sum is equal to the thing.
|
ושרש הסך האמור ישוה הדבר |
|
תמצא לי שני מספרים שיהיה הראשון חלק מהשני כמו שב' הוא מג' ומוכה הראשון בשני יעשה כ' ושרש ח' |
| The procedure: | זהו מעשהו |
|
תניח שהמספר ראשון יהיה ב' דברים |
|
והשני יהיה ג' דברי' |
|
|
עתה תכה הראשון בשני וזהו ב' דברים בג' דברי' |
|
|
ויהיו ו' צינסי שהם שוים אל כ' ושרש ח' |
|
עתה תרדוף כפי הכלל הנתון למעלה |
| תחלק המספרים אשר לא נקבו להיות להם שרש והם כ' על כמות הצינסי שהם ו' שעולה מזה ג' וא' צינסו ושמרם | |
| אח"כ תכה כמות הצינסי בעצמם והם ו' ועולה ל"ו | |
| ותחלק המספרי' הנקובי' להיות להם שרש שזהו ח' על ל"ו שעולה מזה ב' תשיעיות | |
| ושרש אלו הב' תשיעיות תחבר עם אלו הג' וא' שליש שהוא מה שבא מהחלוק מהמספרי' על כמות הצינסי ויהיה לך שרש מחבור ג' ושליש עם שרש ב' תשיעיו' וככה ישוה הדבר | |
|
| |
| ואתה הנחת שהמספר ראשון היה ב' דברים א"כ תכה ב' בשרש חבור ג' ושליש עם שרש ב' תשיעיו' ועולה שרש מחבור י"ג ושליש עם שרש ג' וה' תשיעיו' וככה יבא להיות המספר הראשון | |
|
| |
| והשני הנחת היותו ג' דברים א"כ תכה ג' בשרש חבור ג' ושליש עם שרש ב' תשיעיו' ועולה שרש מחבור ל' עם שרש מי"ח וככה יבא להיות המספר השני | |
|
| |
Chapter 39 |
פרק ל"ט |
| When numbers are equal to squares and a root of squares:
|
עוד באופן אחר כאשר המספרי' יהיו שוים אל הצינסי ואל שרשי צינסי |
| The numbers should be divided by the number of the squares that are not the radicand. Keep the result. | צריך לחלק המספרי' על כמות הצינסי אשר לא נקבו להיות להם שרש ומה שיעלה מזה תשמור |
| Then, multiply the number of the squares that are not the radicand by itself. | ואח"כ תכה כמות הצינסי אשר לא נקבו להיות להם שרש בעצמם |
| The [number of the] squares that are the radicand should be divided by this product, which is as the square of the squares. | ולחלק הצינסי אשר נקבו להיות להם שרש באותה ההכאה שהיא כרבוע הצינסי |
| Add a quarter of the result to the quotient of the numbers divided by the number of the squares. | ורביע מהעולה מזה תחבר אל מה שעלה מחלוקת המספרי' על כמות הצינסי |
| The root of this sum minus the root of the quarter you added is equal to the thing, and it is a root of a number minus a root of a number.
|
ושרש זה הסך פחות שרש אותו הרביע אשר חברת יבא לשוות הדבר ויהיה שרש מספר פחות שרש מספר |
|
תמצא לי שני מספרי' שהאחד יהיה חלק מהאחר כמו שג' הוא מד' ומוכה הראשון בשרש ח' והשני בעצמו ואותן שתי הכאות יחוברו יחד יעשו מ"ח |
| The procedure: | זה מעשהו |
|
תניח שהמספר ראשון יהיה ג' דברים |
|
והשני ד' דברי' |
|
|
עתה תכה הראשון שהוא ג' דברי' בשרש ח' ועולה שרש מע"ב צינסי |
|
|
אח"כ תכה המספר השני שהוא ד' דברי' בעצמו ועולה י"ו צינסי |
|
וחבר שתי אלו ההכאות יחד |
|
|
שהם י"ו צינסי עם שרש ע"ב צינסי |
|
|
ויהיה לך י"ו צינסי ושרש ע"ב צינסי שוים אל מ"ח |
|
עתה תרדוף כפי הכלל הנתון למעלה |
| תחלק המספרים בכמות הצינסי אשר אינם נקובים להיות להם שרש שהם מ"ח בי"ו ויבא מזה ג' ושמרם | |
| אח"כ תכה כמות הצינסי הבלתי נקובים להיות להם שרש בעצמם וזהו י"ו ועולה רנ"ו | |
| וחלק הצינסי הנקובי' להיות להם שרש וזהו ע"ב על רנ"ו ויבא מזה ט' חלקים מל"ב | |
| עתה תקח הרביע מאלו הט' חלקים מל"ב ויהיה לך חלקים מקכ"ח | |
| ואלו הט' מקכ"ח תחבר על המספר שהוא ג' אשר בא בחלקך המספרי' על הצינסי והוא אשר שמרת ויהיה לך ג' וט' מקכ"ח | |
| ושרש ג' וט' מקכ"ח פחות שרש מאלו הט' מקכ"ח שהוא הרביע ממה שבא אליך בחלקך הצינסי הנקובים להיות להם שרש ברבוע הצינסי הבלתי נקובי' להיות להם שרש ככה יבא לשוות הדבר | |
|
| |
|
|
ואתה הנחת היות המספר ראשון ג' דברי' א"כ תכה ג' בשרש ג' וט' מקכ"ח פחות משרש ט' מקכ"ח ועולה שרש כ"ז ופ"א מקכ"ח פחות שרש מפ"א מקכ"ח וכן הוא המספר הראשון |
|
|
והמספר והשני הנחת היותו ד' דברים א"כ תכה ד' דברים בשרש ג' וט' מקכ"ח פחות שרש מט' מקכ"ח ועולה שרש ממ"ט פחות שרש מא' ושמינית וכן יבא להיות המספר השני |
| The equation can be restored to chapter 4: | ודע שזאת ההשואה אפש' להשיבה אל הפרק הרביעי |
|
|
ויהיה לך י"ו צינסי וכך דברי' כמו שהוא שרש מע"ב שוים אל מ"ח |
since 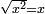
|
מפני כי שרש הצינסו יבא להיות הדבר |
Chapter 40 |
פרק מ' |
| When cubes are equal to numbers and a root of numbers:
|
עוד באופן אחר כאשר המעוקבים יהיו שוים אל מספרים ואל שרשי מספרים |
| The numbers should be divided by the number of the cubes. Keep the result. | צריך לחלק המספר בכמות המעוק' ואשר יבא מזה שמרהו |
| Then, the number of the cubes should be multiplied by itself. | אח"כ יוכו כמות המעוקבי' בעצמם |
| Divide the numbers that are the radicand by this product. | ותחלק המספרי' הנקובי' להיות להם שרש באותה ההכאה |
| Add the root of the result to the quotient of the numbers that are not the radicand divided by the product of [the number of] the cubes. | ושרש העולה מזה תחבר אל מה שעלה מחלוקת המספרי' הבלתי נקובי' היות להם שרש בכמות המעוקבי' |
| The cube root of this sum is equal to the thing.
|
ושרש מעו' מאותו הסך יבא לשוות הדברי' |
|
עשה לי החשבון תמצא לי שני מספרים שיהיה המספר ראשון חלק מהאחר כמו שב' הוא מג' ובהכות הראשון בשני ואותה ההכאה תוכה בשני יעשה מאה ושרש ח' |
| The procedure: | זהו מעשהו |
|
תניח שהמספר הראשון יהיה ב' דברים |
|
והאחר יבא להיות ג' דברי' |
|
|
עתה תכה הראשון בשני וזהו ב' דברי' בג' דברים ועולה ו' צינסי אח"כ תכה זאת ההכאה שהיא ו' צינסי במספר השני שהוא ג' דברי' |
|
|
ועולה י"ח מעוקבי' והם שוים אל ק' ושרש ח' |
|
עתה תרדוף כפי הכלל הנתון למעלה |
|
|
תחלק המספר בכמות המעוקבי' וזהו ק' בי"ח ועולה מזה ה' וה' תשיעיות ושמרם |
| אח"כ תכה כמות המעוקבי' בעצמם והם י"ח שכ"ד | |
| ותחלק המספרי' הנקובי' שהם ח' בשכ"ד ועולה מזה ב' חלקים מפ"א | |
| ושרש ב' מפ"א תחבר אל מה שעלה בחלוקת המספרי' אשר לא נקבו להיות להם שרש בכמות המעוקבים שזהו על ה' וה' תשיעיות ועולה ה' וה' תשיעיות ושרש ב' מפ"א | |
|
|
ואתה הנחת היות המספר ראשון ב' דברי' א"כ תכה ב' בשרש מעוקב מה' וה' תשיעיות מחובר עמם שרש ב' שמיניות |
|
ותזכור שהנך צריך להשיב ב' אל שרש מעוק' ויהיה לך שרש מעוק' מח' |
| ותכה בשרש מעוק' מה' וה' תשיעיו' וזהו שרש ב' מפ"א עולה שרש מעוק' ממ"ד וד' תשיעיות | |
|
|
והשני הנחת היותו ג' דברים א"כ תכה ג' בשרש מעוק' מה' וה' תשיעיו' מחובר עמם שרש ב' מפ"א |
|
ותזכור כי הנך צריך להשיב ג' אל שרש מעו' ויהיה לך שרש מעוק' מכ"ז |
| עתה תכה כ"ז בה' וה' תשיעיות ועולה ק"נ | |
| עתה תכה כ"ז בעצמם ועולה תשכ"ט | |
| ותכה תשכ"ט בב' מפ"א עולה י"ח וזה הי"ח הוא שרש מי"ח |
Chapter 41 |
פרק מ"א |
| When numbers are equal to cubes and a root of cubes:
|
כאשר המספרי' יהיו שוים אל המעוקבי' ושרשי מעוקבי' |
| The numbers should be divided by the number of the cubes that are not the radicand. Keep the result. | צריך לחלק המספרי' בכמות המעוקבי' אשר לא נקבו להיות להם שרש ומה שיבא מזה תשמור |
| Then, multiply [the number of] the cubes that are not the radicand by itself. | אח"כ תכה המעו' אשר לא נקבו להיות להם שרש בעצמם |
| Divide the number of the cubes that are the radicand by this product. | ותחלק כמות המעוק' הנקובי' להיות להם שרש באותה ההכאה |
| [Add] a quarter of the result to the quotient you kept. | ורובע העולה מזה תחלק על החלוק אשר שמרת |
| The root of this sum minus the root of the quarter you added - meaning the root of a quarter of the quotient of the [number of] cubes that are the radicand divided by the product of the [number of] cubes that are not the radicand - is equal to the root of the cube. | ושרש אותו הסך פחות שרש אותו הרובע אשר חברת רצו' שרש הא' רביע ממה שעלה בחלוק המעוק' שנקבו להיות להם שרש בהכאת המעוק' אשר לא נקבו היות להם שרש יבא להיות
שרש המעוק' |
| Multiply this root by itself; the result is the cube. | ותכה זה השרש בעצמו יבא להיות המעוקב |
| The cube root of this product is the thing.
|
ושרש המעו' מזאת ההכאה יבא להיות הדבר |
|
עשה לי זה החשבון תמצא לי שני מספרי' שיהיה הראשון חלק מהשני כמו שב' הוא מג' ומוכה הראשון בעצמו ואותה ההכאה תכה בשני ותחובר זאת ההכאה עם שרשה יעשה שמ"ב |
| The procedure: | זהו מעשהו |
|
תניח שהמספר ראשון יהיה ב' דברי' |
|
והאחר מחוייב שיהיה ג' דברי' |
|
|
עתה תכה המספר הראשון שהוא ב' דברי' בעצמו ועולה ד' צינסי ואלו ד' צינסי תכם על המספר השני שזהו על ג' דברי' |
|
|
ועולה י"ב מעוקבי' ושרש י"ב מעוק' יבאו להיות שוים אל שמ"ב |
|
עתה תרדוף כפי הכלל הנתון למעלה |
|
|
תחלק המספרי' בכמות המעוק' שזהו שמ"ב על י"ב ויבא מזה כ"ח וחצי ושמרם |
| אח"כ תכה כמות המעוק' אשר לא נקבו להיות להם שרש והם י"ב בעצמם ועולה קמ"ד | |
| ותחלק כמות המעוקבי' הנקובי' להיות להם שרש שהם הי"ב האחד בקמ"ד ויעלה מזה א' מי"ב | |
| ותקח הרביע מזה הא' חלק מי"ב שהוא א' חלק ממ"ח | |
| ותחברהו עם כ"ח וחצי אשר שמרת ויהיה לך כ"ח וכ"ה ממ"ח | |
| ושרש כ"ח וכ"ה ממ"ח פחות שרש אותו הרביע דהיינו פחות שרש א' ממ"ח יבא להיות שרש המעוקב | |
| ותכה זה השרש בעצמו רצוני שרש כ"ח וכ"ה ממ"ח פחות שרש א' ממ"ח בעצמו ועולה כ"ז וככה יבא להיות המעוק' | |
|
|
ואתה הנחת היות המספר ראשון ב' דברי' א"כ תכה ב' במה ששוה הדבר וזהו ג' ועולה ו' וכן יבא להיות המספר ראשון |
|
|
והשני הנחת היותו ג' דברי' א"כ תכה ג' בג' ועולה ט' וככה יבא להיות המספר השני |
Chapter 42 |
פרק מ"ב |
| When squares of squares are equal to a number and a root of a number:
|
עוד כאשר הצינסי מצינסי יהיו שוים אל מספר ושרש מספר |
| The numbers should be divided by the number of the squares of squares. Keep the result. | צריך לחלק המספרי' בכמות הצינסי מצינסי ומה שיעלה ישמור |
| Then, the number of the squares of squares should be multiplied by itself. | ואח"כ יוכה כמות צינסי מצינסי בעצמו |
| The numbers that are the radicand should be divided by this product. | ולחלק המספרי' הנקובי' להיות להם שרש באותה ההכאה |
| The root of the result is added to the quotient we kept, which is the result of division of the numbers that are not the radicand by the number of the squares of squares. | ושרש מה שיבא מזה יחובר אל החלוק אשר שמרנו וזהו מה שבא בחלוק המספרי' אשר לא נקבו היות להם שרש בכמות הצינסי מצינסי |
| The root of the root of this sum is equal to the thing.
|
ושרש השרש מאותו הסך יבא לשוות הדבר |
|
עשה לי זה החשבון שיהיה האחד חלק מהאחר כמו שב' הוא חלק מג' ובהכות הראשון בשני ואותה ההכאה תוכה בעצמה יעשה כ' ושרש ל' |
| The procedure: | זהו מעשהו |
|
תניח שהמספר ראשון יהיה ב' דברי' והשני ג' דברים |
|
|
עתה תכה הראשון בשני וזהו ב' בג' ועולה ו' צינסי ואלו הו' צינסי תכם בעצמם |
|
|
ויעלו ל"ו צינסי מצינסי אשר הם שוים אל כ' ושרש ל' |
|
עתה תרדוף כפי הכלל האמור למעלה |
|
|
תחלק המספרי' על כמות הצינסי מצינסי שזהו כ' על ל"ו שבא מזה ה' תשיעיות ושמרם |
| אח"כ תכה כמות הצינסי מצינסי בעצמם וזהו ל"ו ועולה אלף רצ"ו | |
| ותחלק המספרי' הנקובי' להיות להם שרש שהם ל' על אלף ורצ"ו ועולה מזה ה' מרי"ו | |
| ושרש אלו הרי"ו תחבר אל החלוק אשר שמרת שהוא על ה' תשיעיות ויהיה לך ה' תשיעיות ושרש ה' מרי"ו | |
|
|
ואתה הנחת היות המספר ראשון ב' דברים א"כ תכה ב' בשרש שרש ה' מט' מחובר עם שרש ה' מרי"ו |
|
|
והשני הנחת היותו ג' דברי' א"כ תכה ג' בשרש שרש ה' מט' מחובר עם שרש ה' מרי"ו |
Chapter 43 |
פרק מ"ג |
| When numbers are equal to squares of squares and a root of squares of squares:
|
כאשר המספרי' יהיו שוים אל הצינסי מצינסי ואל שרשי צינסי מצינסי |
| The numbers should be divided by the number of the squares of squares that are not the radicand. Keep the result. | צריך לחלק המספרי' על כמות הצינסי מצינסי אשר לא נקבו להיות להם שרש ומה שיבא מזה תשמור |
| Then, multiply the number of the squares of squares that are not the radicand by itself. | ואח"כ תכה כמות הצינסי מצינסי אשר לא נקבו להיות להם שרש בעצמם |
| The [number of the] squares of squares that are the radicand should be divided by this product. | ולחלוק הצינסי מצינסי הנקובים להיות להם שרש באותה ההכאה |
| Add a quarter of the result to the quotient of the numbers divided by the number of the squares of squares. | ורביע ממה שיבא תחבר אל החלוק שבא לך מהמספרים על כמות הצינסי מצינסי |
| The root of this sum minus the root of the quarter is equal to the square. | ושרש ממרובע מהסך ההוא פחות שרש אותו הרביע אשר יבא לשוות הצינסו |
| The root of this remainder is equal to the thing; that is the root of the quarter subtracted from the root of the sum and the root of the remainder is equal to the thing.
|
ושרש זה השארית יבא לשוות הדבר וזהו שרש אותו הרביע מוצא משרש הסך שרש הנשאר יבא להיות שוה הדבר |
|
עשה לי זה החשבון תמצא לי שני מספרי' שיהיה הא' חלק מהאחר כמו שב' הוא מג' ומוכה הראשון בשני וזאת ההכאה תוכה בעצמה ותחובר זאת ההכאה עם הכאת הראשון מוכה בעצמו ומה שיבא בשרש ד' יעשה ד' ורביע |
| The procedure: | זהו מעשהו |
|
תניח שהמספר הראשון יהיה ב' דברי' והשני יהיה ג' דברים |
|
|
עתה תכה הראשון בשני וזהו ב' דברים בג' דברי' ועולה ו' צינסי וזאת ההכאה שהוא ו' צינסי תכה בעצמה ועולה ל"ו צינסי מצינסי ותשמרם |
|
|
אח"כ תכה המספר ראשון שהוא ב' דברים בעצמו ועולה ד' צינסי ואלו הד' צינסי תכה בשרש ד' ועולה שרש מס"ד צינסי מצינסי |
|
|
עתה תחבר שרש מס"ד צינסי מצינסי עם מה ששמרת שזהו עם ל"ו צינסי מצינסי |
|
|
ויהיה לך ל"ו צינסי מצינסי ושרש מס"ד צינסי מצינסי היות שוים אל ד' ורביע |
|
עתה תרדוף כפי הכלל האמור למעלה |
|
|
תחלק המספרים על כמות הצינסי מצינסי אשר לא נקבו היות להם שרש שזהו ד' ורביע בל"ו ויבא מזה י"ז מקמ"ד ותשמרם |
| אח"כ תכה כמות הצינסי מצינסי אשר לא נקבו היות להם שרש בעצמם וזהו ל"ו ועולה אלף ורצ"ו | |
| ותחלק הצינסי מצינסי הנקובי' להיות להם שרש שזהו ס"ד באלף ורצ"ו ויבא מזה ד' מפ"א | |
| עתה תקח הרביע מאלו הד' מפ"א ויהיה לך א' מפ"א | |
| תחברהו על י"ז מקמ"ד שבאו בחלוקת המספרים על כמות הצינסי מצינסי וזהו אשר שמרת ויהיה לך קס"ט מאלף ורצ"ו | |
| ושרש מקס"ט מאלף ורצ"ו פחות שרש מזה הא' מפ"א שזהו הרביע ממה שבא לך בחלקך הצינסי מהצינסי הנקובים להיות להם שרש על הכאת הצינסי מצינסי אשר לא נקבו להיות להם שרש | |
|
|
ואתה הנחת שהמספר ראשון היה ב' דברים א"כ תכה ב' בשרש השארית הנשאר מהוצאת שרש א' מפ"א חוץ משרש קס"ט מאלף רצ"ו |
|
ותזכור כי הנך צריך להשיב ב' אל שרש משרש ויהיה לך שרש משרש י"ו |
| ותכה אלו הי"ו בקס"ט מאלף ורצ"ו ועולה אלפיים ותש"ד מאלף ורצ"ו שהם ב' וז' מפ"א ושרש מזה תשמור | |
| ואח"כ תכה י"ו בא' מפ"א ועולה י"ו מפ"א | |
| ושרש זה הי"ו מפ"א תוציא מהשרש אשר שמרת שהוא מב' וז' מפ"א | |
| ושרש הנשאר יבא להיות המספר ראשון | |
| ואם רצית להשיבו אל שלמים דע כי שרש ב' וז' תשיעיות הוא א' וד' מט' | |
|
|
והשני הנחת היותו ג' דברי' א"כ תכה ג' במה ששוה הדבר |
| This calculation can be restored to another chapter | ודע כי זה החשבון היה יכול לבוא אל פרק אחר |
|
אם היית מכה הכאת המספר הראשון שהיתה ד' צינסי בב' שהוא שרש ד' |
|
אבל ברצותנו להשיבו אל זה הפרק הוכה בשרש ד' כאלו לא היה אל ד' שרש מדבר |
Chapter 44 |
פרק מ"ד |
| עוד באופן אחר | |
| When squares of squares plus squares are equal to a number:
|
כאשר הצינסי מצינסי וצינסי יהיו שוים אל מספר |
| The whole equation should be divided by the number of the squares of the squares. | צריך לחלק כל ההשואה על כמות הצינסי מצינסי |
| Then, the number of the squares should be halved. | ואח"כ לחלק כמות הצינסי לחצי |
| [One] half should be multiplied by itself. | ולהכות כל אחד מהחצאים בעצמו |
| Add the product to the number. | והעולה מזה תחבר על המספר |
| Subtract the other half of the number of the squares from the root of the sum. | ומשרש הסך תוציא המחצית האחר מכמות הצינסי |
| The root of the remainder is equal to the thing.
|
ושרש הנשאר יבא לשוות הדבר |
|
ואשים לך המשל ואומ' כן עשה לי זה החשבון תמצא לי שני מספרי' שכאשר יוכה הראשון בעצמו יעשה השני פחות ה' ויוכו כל אחד מהם בעצמו ויחוברו אותם ההכאות יחד יעשה ק' |
|
זהו מעשהו |
|
תניח שהמספר הראשון יהיה דבר אחד |
|
ותכהו בעצמו והיה א' צינסו |
|
א"כ יבא להיות השני א' צינסו וה' מספרים |
|
עתה תכה כל אחד מהם בעצמו |
|
ועולה הראשון שהוא דבר אחד א' צינסו |
|
והשני שהוא א' צינסו וה' מספרים עולה הכאתו בעצמו א' צינסו מצינסו וי' צינסי וכ"ה מספרי' |
|
ותחבר שתי אלו ההכאות יחד ויהיה לך א' צינסו מצינסו וי"א צינסי וכ"ה מספרים אשר יבאו להיות שוים אל ק' |
|
עתה הוצא המספרים שהם פוחתים בכמות מאחד מהחלקים מכל אחד מהחלקים |
|
וישאר אחד מהחלקים א' צינסו מצינסו וי"א צינסי בלתי מספר שוה לחלק האחר אשר ישאר ע"ה מספרים |
|
עתה תרדוף כפי הכלל האמור למעלה |
|
תחלק כל ההשואה על כמות הצינסי מצינסי שהוא אחד ויבא מזה ההשואה ההיא בעצמה |
| אח"כ תחלק כמות הצינסי לחצי וזהו י"א ויבא מזה ה' וחצי | |
| ואכה אלו הה' וחצי בעצמם ועולה ל' ורביע | |
| וחברם על המספרים שזהו על ע"ה ויהיה לך ק"ה ורביע | |
| ומשרש אלו הק"ה ורביע הוצא מחצית הצינסי שהוא ה' וחצי וישאר שרש ק"ה ורביע פחות ה' וחצי | |
| ושרש זה הנשאר יבא להיות הדבר וזהו המספר הראשון | |
|
| |
| עתה תכה שרש זה השארית בעצמו וזהו שרש מה' וחצי משרש ק"ה ורביע בשרש מה' וחצי משרש ק"ה ורביע ועולה שרש מק"ה ורביע פחות ה' וחצי | |
| ועתה אמ' קודם בשאלה שהמספר ראשון מוכה בעצמו ראוי שיעשה השני פחות ה' א"כ יבא לעשות השני ה' יותר מהכאת הראשון | |
| ולכן תוסיף על הכאת הראשון שזהו על שרש ק"ה ורביע פחות ה' וחצי האמור ויהיה לך שרש ק"ה ורביע פחות חצי וכן יבא להיות המספר השני | |
|
| |
Chapter 45 | ||
Chapter 45-I |
פרק מ"ה | |
| עוד אחר באופן אחר | ||
| When squares are equal to squares of squares and a root of a number:
|
כאשר הצינסי יהיו שוים אל הצינסי מצינסי ואל מספר | |
| The whole equation should be divided by the number of the squares of squares. | צריך לחלק כל ההשואה על כמות הצינסי מצינסי | |
| Then, the number of the squares should be halved. | ואח"כ לחלק כמות הצינסי לחצי | |
| One half should be multiplied by itself. | ולהכות אחד מהחצאים על עצמו | |
| Subtract the number from the product. | ומאותה ההכאה תוציא המספרים | |
| Add the root of the remainder to the other half of [the number of] the squares. | ושרש הנשאר תחבר על המחצית האחר מהצינסי | |
| The root of the sum is the thing.
|
ושרש אותו הסך יבא להיות הדבר | |
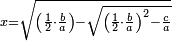
|
וחשבונות מה צריכי' לענות שהדבר ישוה שרש הנשאר בהוציא המספרי' מהכאת מחצית כמות הצינסי ושרש הנשאר ההוא יוציא מהחצי האחר מהצינסי | |
| In the first answer the root of the remainder is added to half the squares, and the root of the sum is the result. | וזהו כמו שבתשובה הראשונה יחובר שרש הנשאר על החצי האחר מהצינסי ושרש הסך ההוא יבא להיות הדבר | |
| Likewise, the root of the remainder is subtracted from half the squares, and the root of this remainder is the result. | כמו כן יוצא להפך שרש מאותו שנשאר ממחצית כמות הצינסי ושרש אותו השארית יבא לשוות הדבר | |
| Many calculations can be solved by these procedures | וחשבונות רבים אפש' לענות בם היות הדבר כמו שכל אחד מהאופנים אומר | |
| Example: | והנני אשים לך משל לפניך כמו שתוכל לראות לפנים | |
|
עשה לי זה החשבון תמצא לי שני מספרי' שיהיה הראשון חלק מהשני כמו שג' הוא מד' ומוכה הראשון בשני ואותה ההכאה תוכה בעצמה ויחובר עמה כ"ז יעשה המספר השני בכפלו בעצמו ואותה ההכאה תוכה בט' | |
| The procedure: | זהו מעשהו | |
|
תניח שהמספר ראשון יהיה ג' דברים והשני יהיה ד' דברים | |
|
|
עתה תכה הראשון בשני ועולה י"ב צינסי ואותה ההכאה מוכה בעצמה ועולה קמ"ד צינסי מצינסי | |
|
|
אח"כ תכה המספר השני שהוא ד' דברים בעצמו ועולה י"ו צינסי וזאת ההכאה תכה בט' | |
|
|
ועולה קמ"ד צינסי אשר יבאו להיות שוים אל החלק האחר אשר שמרת וזהו אל קמ"ד צינסי מצינסי וכ"ז מספרי' | |
|
תחלק כל ההשואה על כמות הצינסי מצינסי שזהו על קמ"ד | |
|
|
ויהיה לך א' צינסו מצינסו וכ"ז מקמ"ד מספרים שוים אל א' צינסו | |
|
|
עתה תחלק כמות הצינסו שהוא א' לחצי ויהיה לך חצי אחד ותכהו בעצמו ועולה א' רביע | |
| ואתה הנחת היות המספר ראשון ג' דברי' א"כ
תכה ג' בשרש מחבור שרש ט' מקמ"ד עם חצי | ||
|
| ||
| והשני הנחת היותו ד' דברים א"כ תכה ד' בשרש מחבור שרש ט' מקמ"ד עם חצי | ||
|
| ||
| The answer of this calculation is by the adding the root to half the squares. | ודע כי זה החשבון נעשית התשובה באופן השרש שיתחבר עם חצי הצינסי | |
| It can be solved also by subtracting the root from half the squares: | ואפש' לענותו ג"כ באופן שיוצא השרש ממחצית הצינסי | |
| באופן זה שכאשר הוצאת המספרי' מהכאת מחצית הצינסי ישאר לך ט' מקמ"ד ושרש זה הט' מקמ"ד בתשובה הראשונה חברת על מחצית הצינסי שהיה חצי ונשאר פחות שרש ט' מקמ"ד | ||
|
| ||
| א"כ הדבר אשר הנחת היותו ג' דברים יבא להיות ג' מוכה בשרש ממוצא שרש מט' מקמ"ד חוצה מחצי שעולה שרש ממוצא שרש מה' וט' מקמ"ד חוצה מד' וחצי וככה יבא להיות המספר הראשון | ||
|
| ||
| והשני הנחת היותו ד' דברים א"כ תכה ד' בשרש ממוצא שרש מט' מקמ"ד חוץ מחצי | ||
|
| ||
| So, the problem is solved by both the above mentioned procedures | והנה נענה זה החשבון בשני האופנים האמורי' למעלה | |
| There are problems that can be solved by both procedures, and both are correct, as seen in this problem. | שאפש' בחשבונו' מה לענות בשני האופני' באמת כן באחד כמו בשני כאשר הראית בזה החשבון | |
Chapter 45 - II |
פרק מ"ה |
| There are problems that can be solved only by one of the procedures of the above chapter: | עוד רצוני להראותך איך הפרק האמור אי אפש' ליתן תשובה בחשבונות מה רק באחד מהאופני' האמורים כמו שאראך מכאן ולהבא בזה החשבון הנמשך |
|
עשה לי זה החשבון תחלק עשרה לשני חלקים באופן שמוכה ההבדל שביניהם בעצמו ואותה ההכאה תוכה בז' ותשיעית יעשה כמו מוכה הראשון בשני ואותה ההכאה תוכה בעצמה אשאל כמה יבא להיות כל אחד מהחלקים |
| The procedure: | זהו מעשהו |
|
תניח שאחד מהחלקים יהיה דבר אחד וחמשה והאחר יבא להיות השארית עד עשרה שהוא ה' פחות דבר אחד |
| עתה תקח ההבדל אשר בין האחד החלק אל האחר אשר יבא להיות ב' דברים מפני שהחלק הגדול הוא ב' דברים יותר מהשני | |
|
| |
|
|
עתה תכה הראשון בשני שזהו דבר אחד וה' בה' פחות דבר אחד ועולה כ"ה מספרי' פחות א' צינסי ואלו כ"ה מספרים פחות א' צינסו תכם בעצמם |
|
|
ועולה א' צינסו מצינסו ותרכ"ה מספרי' פחות נ' צינסי שהם שוים אל החלק האחר אשר שמרת שזהו אל כ"ח צינסי וד' תשיעיות |
|
|
עתה תחבר נ' צינסי הפוחת מהחלק האחד אל כל אחד מהחלקים |
|
|
ויהיה לך א' צינסו מצינסו ותרכ"ה מספרים שוים אל תשפ"ט צינסי וד' מט' |
|
עתה תרדוף כפי הכלל האמור למעלה |
|
תחלק כל ההשואה על כמות הצינסי מצינסי שזהו א' ויהיה לך אותו בעצמו |
| אח"כ תחלק כמות הצינסי לחצי שזהו ע"ח וד' תשיעיות ויעלה מזה ל"ט וב' מט' | |
| ואלו הל"ט וב' מט' תכה בעצמם ועולה אלף תקל"ח ול"א מפ"א | |
| ומזאת ההכאה תוציא המספרים שהם תרכ"ה וישאר תתקי"ג ול"א מפ"א | |
| ושרש זה הנשאר תוציא חוצה מהחצי האחד מהצינסי שזהו חוץ מל"ט וב' מט' ויהיה לך ל"ט וב' מט' פחות שרש תתקי"ג ול"א מפ"א ושרש זה הנשאר יבא לשוות הדבר | |
|
| |
|
|
ואתה הנחת שאחד מהחלקים היה דבר אחד וה' א"כ יבא להיות החלק הראשון ה' ומוצא שרש מתתקי"ג ול"א מפ"א חוץ מל"ט וב' מט' |
|
|
והחלק האחר יבא להיות ה' פחות הדבר האמור שהוא פחות שרש ממוצא שרש מתתקי"ג ול"א מפ"א חוץ מל"ט וב' מט' וככה יבא להיות החלק האחר מעשרה |
| This is solved only by one of the solving procedures mentioned above, which is by subtracting: | עתה אזכירך שהדבר נענה באופן אחד מהאופנים שהוא באופן מההוצאה האמור קודם בכלל האמור בפרק האמור |
| שהוא שהדבר יבא להיות שרש ממוצא מתתקי"ג ול"א מפ"א שהוא ל' וב' מט' חוץ מל"ט וב' מט' וישאר ט' | |
|
| |
|
|
והחלק השני הוא ה' פחות דבר אחד והדבר הוא ג' שישאר ב' וכן הוא החלק השני |
| It cannot be solved by the other procedure of the above chapter. | ובאופן אחר אי אפש' לענות הדבר בהשיב זה החשבון אל זה הפרק |
Since one of the part is 
|
מפני כי אחד מהחלקים יבא להיות דבר אחד וה' |
|
|
ובזולת אותו השרש מהנשאר בהוצאת המספרי' מהכאת מחצית כמות הצינסי לבד השרש ממחצית כמות הצינסי יבא להיות יותר מה' |
|
|
א"כ יבא להיו' אחד מהחלקי' ה' ודבר אחד והדבר יבא להיות יותר מה' באופן שהחלק האמור יבא להיות יותר מעשרה |
| and it is impossible that the part will be greater than the whole | וזה דבר נמנע שהחלק יהיה גדול מהכל |
Chapter 45 - III |
פרק מ"ה |
| Sometimes the answer is given only by the other procedure of the above chapter, i.e. the root of the sum. | עוד רצוני להשים לפניך חשבון אחר אשר עמו יראה כי הפרק האמור יתן תשובה לפעמים היות הדבר לבד באופן האמור קודם רצו' שרש החבור |
| Example: | ואתן לך המשל פה בקרוב באופן זה כאמור |
|
עשה לי מעשרה ב' חלקים באופן שבהכות הגדול בקטן ויחובר אל הכאת הגדול בעצמו ואותו הסך יוכה בחלק הגדול יעשה כמו הכאת הגדול בחציו ואותה ההכאה תוכה בעצמה ויחובר אליה ל"ו אשאל כמה יבא להיות כל אחד מהחלקי' |
| The procedure: | זו היא פעולתו |
|
תניח שהחלק הגדול יהיה דבר אחד והקטן יבא להיות הנשאר עד עשרה שהוא עשרה פחות דבר |
|
|
עתה תכה הגדול בקטן רצו' דבר אחד בעשרה פחות דבר אחד ועולה י' דברי' פחות א' צינסו |
|
|
ועל זה תחבר הכאת הגדול המוכה בעצמו שהוא דבר אחד בדבר אחד ועולה א' צינסו ויהיה לך בסך עשרה דברים |
|
|
ותכה זה הסך שהוא י' דברי' בחלק הגדול שהוא דבר אחד ועולה י' צינסי ושמרם לחלק אחד מההשואה |
|
|
אח"כ תכה הגדול שהוא א' דבר בחציו שהוא חצי דבר ועולה חצי צינסו |
|
|
וזאת ההכאה רצוני חצי צינסו תכה בעצמה |
|
|
ועולה א' רביע מצינסו מצינסו ול"ו מספרי' להיות שוים אל עשרה צינסי אשר שמרת |
|
עתה תרדוף כפי הכלל האמור למעלה |
|
תחלק כל ההשוא' בכמות הצינסי מצינסי שהוא א' רביע |
|
|
ויהיה לך א' צינסו מצינסו וקמ"ד מספרי' שוים אל מ' צינסי |
| אח"כ תחלק כמות הצינסי שהם מ' לחצאי' ויבא מזה כ' ואלו הכ' תכם בעצמם ועולה ת' | |
|
| |
|
|
והחלק האחר יבא להיות הנשאר עד י' וזהו עשרה פחות שרש מחבור שרש רנ"ו על הכ' שהם מחצית כמות הצינסי |
| It is solved by the first procedure of the [above] chapter, i.e. the root of the sum, but it cannot be solved by the other procedure. | ונענה באופן ראשון שמשים הפרק שזהו שרש החבור ולא יתכן תשובתו באחד מהאופני' האחרי' |
|
וסבת למה זה היא כי כשהוצא שרש רנ"ו ממחצית הצינסי שזהו הכ' שרש הנשאר יבא להיות פחות ממחצית עשרה |
|
וכבר אמרנו קודם שהחלק הגדול היה דבר אחד ולעשות מעשרה שני חלקי' |
|
ולקחת הגדול הנה הגדול יבא להיות יותר מה' וכפי זה החשבון היה בא החלק הגדול פחו' מה' וזהו דבר נמנע שיהיה החלק מי' פחות מה' |
| Therefore:
|
א"כ תחבר שרש רנ"ו שהוא י"ו על כ' עולה ל"ו ושרש זה הסך מל"ו שיבא להיות ו' הוא החלק ראשון אשר הונח היותו דבר אחד |
|
|
והחלק הקטן יבא להיות הנשאר עד י' שהוא ד' |
The reason that the larger part was given as  , was to solve it by the third way of the above chapter, i.e. to show that sometimes it is impossible to solve by the other procedure, only by the root of the sum. , was to solve it by the third way of the above chapter, i.e. to show that sometimes it is impossible to solve by the other procedure, only by the root of the sum.
|
עתה דע כי הסבה שהנחנו החלק הגדול דבר א' היתה כדי להשיב תשובת זה הפרק אל התשוב' השלישית אשר הונחה בראשית הפרק ולהראות כי בפרקי' מה הדבר אי אפש' לענות באופן אחר כי אם שרש הסך כאשר אמרנו קודם |
Chapter 46 |
פרק מ"ו |
| עוד רצוני לרדוף וזהו הוא בזה האופן | |
| When squares of squares are equal to a number and squares:
|
כאשר הצינסי מצינסי יהיו שוים אל המספר והצינסי |
| The whole equation should be divided by the number of the squares of squares. | צריך לחלק כל ההשואה על כמות הצינסי מצינסי |
| Then, the number of the squares should be divided in half. | ואח"כ לחלק כמות הצינסי לחצאי' |
| Each of these halves is divided by itself. | ולהכות אחד מהחצאי' ההם בעצמו |
| Add the numbers to this product. | ועל זאת ההכא' תחבר המספרי' |
| Add the root of this sum to the other half of the number of squares. | ושרש זה הסך תחבר על החצי האחר מכמות הצינסי |
| The root of this final sum is equal to the thing.
|
ושרש זה הסך האחרון יבא לשוו' הדבר |
|
והנה המשל עשה לי זה החשבון תמצא לי ב' מספרי' שכאשר יוכה הראשון בעצמו יעשה ב' דמיוני השני וח' יותר ומוכה כל אחד מהם בעצמו ויקובצו ב' ההכאו' יחד יעשו פ' |
|
תניח שהמספר הראשון יהיה דבר אחד |
|
וזה תכהו בעצמו ועולה א' צינסו |
|
א"כ יבא להיות המספר השני חצי צינסו פחות ד' |
|
עתה תכה כל אחד מהם בעצמו |
|
שהם הראשון שהוא דבר אחד ועולה א' צינסו |
|
והשני שהוא חצי צינסו פחות ד' בעצמו ועולה א' רביע מצינסו מצינסו וי"ו מספרי' פחות ד' צינסו |
|
וקבץ ב' אלו ההכאו' יחד |
|
וזהו א' צינסו עם רביע צינסו מצינסו וי"ו מספרי' פחות ד' צינסי |
|
עולה א' רביע צינסו מצינסו וי"ו מספרי' פחות ג' צינסי שהם שוים אל פ' |
|
עתה תרדוף כפי הכלל הנתון למעלה |
|
תחלק כל ההשואה על כמו' הצינסי מצינסי וזהו על א' ורביע |
|
וקודם שתחלק זאת ההשוא' תתן לאשר לו פחות א' מהחלקי' שהוא ג' צינסי אל כל א' מהחלקי' והוצא הכמות הקטן מהמספרי' מכל א' מהחלקי' |
|
וישאר לך ההשוא' נקיה א' ורביע צינסו מצינסו שוה אל ג' צנסי ואל ס"ד מספרי' |
|
לחלק על א' רביע |
|
ויבא מזה א' צינסו מצינסו שוה אל י"ב צינסי ורנ"ו מספרי' |
| אח"כ תחלק כמות הצינסי שהם י"ב לחצי ויבא ו' ואלו הו' תכם בעצמם ועולה ל"ו | |
|
| |
| עתה תכה זה שיבא להיות הדבר בעצמו ויהיה לך ו' ושרש רצ"ב ומזה הסך תוציא ח' וישאר שרש מרצ"ב פחות ב' אשר יבא להיות כפל למספר השני | |
|
| |
Chapter 47 |
פרק מ"ז |
| When things are equal to a cube root of the numbers:
|
כאשר הדברי' יהיו שוי' אל שרש מעוק' ממספרי' |
| The number of the things should be cubed. | צריך להשיב כמות הדברי' אל מעוק' |
| Then, the numbers that are the radicand of the cube root should be divided by the cubed number of the things. | ואח"כ לחלק המספרי' הנקובי' להיות להם שרש מעוק' על השבת כמות הדברי' |
| Extract the cube root of the result and it is equal to the thing.
|
ומהעולה תקח שרשו המעו' יבא לשוות הדבר |
|
והנה המשל תמצא לי ב' מספרי' שיהיה האחד חלק מהאחר כמו שב' הוא מג' ומוכה הראשון בג' והשני בד' ויחוברו ב' אלו ההכאו' יחד יעשה שרש מעוק' מרי"ו |
|
תעשה כמו שאומ' הכלל שלו |
|
תניח שהמספר הראשון יהיה ב' דברי' והשני ג' |
|
עתה תכה הראשון שהוא ב' דברי' בג' ועולה ו' |
|
אח"כ תכה השני שהוא ג' דברי' בד' ועולה י"ב דברי' |
|
ותקבץ שתי אלו ההכאו' יחד רצו' ו' דברי' עם י"ב דברי' ועולה י"ח דברי' שהם שוים אל שרש מעוק' מרי"ו |
|
עתה תרדוף כפי הכלל הנתון למעלה |
|
תשיב כמות הדברי' אל מעוק' וזהו י"ח בזה האופן תכה י"ח בעצמו ועולה שכ"ד ואלו השכ"ד תכם בי"ח ועולה ה' אלפי' ותתל"ב |
|
ותחלק כמות המספרי' אשר להם שרש מעו' שהם רי"ו כ"ה אלפי' ותתל"ב ויבא מזה א' מס' |
|
ושרש מעו' מא' מכ"ז יבא להיות הדבר |
|
ואתה הנחת היות המספר הראשון ב' דברי' א"כ תכה ב' בשרש מעו' מא' מכ"ז ועולה שרש מעו' מח' מכ"ז אשר יבא להיות ב' שלישים וככה יבא להיות המספר הראשו' |
|
והשני הנחת היותו ג' דברי' א"כ תכה ג' בשרש מעו' מא' מכ"ז ועלה שרש מכ"ז שהוא אחד שלם שהוא א' וכך יהיה המספר השני |
| [The equation] can be returned to chapter 7, by cubing each [part of the equation] by itself. | ואפשר להשיב אל פרק ז' בהכות כל אחד בעצמו באופן מעו' |
Chapter 48 |
פרק מ"ח |
| עוד רצו' לשים לפניך חשבון אחר באופן אחר ואומ' כן | |
| When numbers are equal to a cube root of a thing:
|
כאשר המספרי' יהיו שוים אל שרש מעו' מדבר |
| The numbers should be cubed. | צריך להשיב כמות המספרי' אל מעו' |
| Then the cubed [numbers] should be divided by the number of the things that are the radicand of the cube root. | ואח"כ לחלק אותה ההשבה על כמות הדברי' הנקובי' להיות להם שרש מעו' |
|
ומה שיבא מזה שוה הדבר |
|
והנה המשל עשה לי זה החשבון תמצא לי ב' מספרי'
שיהיה הא' חלק מהאחר כמו שג' הוא מד' |
|
זו היא פעולתו |
|
תניח שהמספר הראשון יהיה ג' דברי' והשני יהיה ד' |
|
עתה תכה הראשון שהוא ג' בב' עולה ו' דברי' |
|
והשני שהוא ד' דברי' תכה בג' ועולה י"ב |
|
ותקבץ שתי אלו הכאות יחד שהם ו' דברי' עם י"ב דברי' ועולה י"ח דברי' |
|
ושרש מעו' מאלו הי"ח דברי' יבא להיות שוה אל ח' |
|
עתה תרדוף כפי הכלל האמור למעלה |
|
אתה צריך להשיב כמות הדברי' אל מעו' ויהיה לך ח' מושב והוא תקי"ב |
|
ותחלק אלו התקי"ב בכמות הדברי' הנקובי' להיות להם שרש מעו' וזהו על י"ח ויבא מזה כ"ח וד' מט' וכך יבא לשוות הדבר |
|
ואתה הנחת היות המספר ראשון ג' דברי' א"כ תכה ג' בכ"ח וד' מט' ועולה פ"ה ושליש וכך יבא להיות המספר ראשון |
|
והשני הנחת היותו ד' דברי' א"כ תכה ד' בכ"ח וד' מט' ועולה קי"ג וז' מט' וכך יבא להיות המספר השני |
| Know that this equation can be returned to the first chapter by cubing this way: | ודע כי זאת ההשוא' אפש' להשיבה אל הפרק הראשו' בהכות עצמו באופן מעו' בזה האופן |
| Multiplying a cube root of a thing by a cube root of a thing generates a cube root of a square.
|
בהכות שרש מעו' מדבר בשרש מעו' מדבר ועושה שרש מעו' מצינסו |
| A cube root of a square by a cube root of a thing generates a cube root of a cube, which is the thing.
|
ושרש מעו' מצינסו בשרש מעו' מדבר יעשה שרש מעו' ממעו' שזהו הדבר |
| Restore the things this way. | וכן תשיב הדברי' |
Chapter 49 |
פרק מ"ט |
| עוד באופן אחר | |
| When squares are equal to a cube root of the numbers:
|
כאשר הצינסי יהיו שוים אל שרשי' מעו' ממספרי' |
| The number of the squares should be cubed. | צריך להשיב כמות הצינסי אל מעוק' |
| Then, the radicand of the cube root is divided by cubed number of the squares. | ואח"כ לחלק המספרי' הנקובי' להיות להם שרש מעו' בהבאת כמות הצינסי אל מעו' |
| The [square] root of a cube root of the result is equal to the thing.
|
ושרש משרש ממעו' והעולה מזה שוה הדבר |
|
והנה המשל תמצא לי ב' מספרי' שיהיה האחד חלק מהאחר כמו ג' מה' ומוכה הראשון בשני יעשה שרש מעו' מתשכ"ט |
|
תניח שהמספר ראשון יהיה ג' דברי' והשני יהיה ה' דברי' |
|
עתה תכה הראשון בשני וזהו ג' דברי' בה' ועולה ט"ו צינסי שהם שוים אל שרש מעו' מתשכ"ט |
|
עתה תרדוף כפי הכלל הנתון למעלה |
|
תשיב כמות הצינסי שהם ט"ו אל מעו' ועולה ג' אלפי' ושע"ה |
|
ותחלק המספרי' הנקובי' להיות להם שרש מעו' שזהו תשכ"ט על ג' אלפי' ושע"ה ויבא מזה כ"ז מקכ"ה |
|
ושרש המרו' משרש המעו' או תאמ' שרש המעו' משרש המרוב' מכ"ז מקכ"ה יבא לשוות הדבר |
|
ואתה הנחת היות המספר ראשון ג' דברי' א"כ תכה ג' בשרש מעו' משרש מרוב' מכ"ז מקכ"ה ועולה שרש מרו' משרש מעוק' מקכ"ה ונ"ח מקכ"ה |
|
והשני הנחת היותו ה' דברי' א"כ תכה ה' בשרש מעו' משרש מרוב' מכ"ז מקכ"ה ועולה שרש מרו' משרש מעו' מג' אלפי' ושע"ה |
| Know that this equation can be returned to chapter 21: | ודע כי זאת ההשואה אפש' להשיב' אל הפרק הכ"א |
| Since the squares [the are equal] to the cube root of the numbers become cubes [that are equal] to the square root of the numbers.
|
מפני כי כך יבאו להיות הצינסי אל שרש מעו' ממספרי' כמו המעו' אל שרש מרוב' ממספרי' |
| Because, by cubing the squares, they becaome squares of squares of square, or cubes of cubes.
|
מפני כי בהשבת הצינסי בהכא' באופן המעו' יעשו צינסי מצינסי מצינסי או מעו' ממעו' |
| Likewise, by squaring the cubes, they become squares of squares of squares, or cubes of cubes.
|
וכדומה לזה יעשה מעו' בהכותו כמו מרוב' צינסי מצינסי מצינסי או מעו' ממעו' |
| Also, a cubed square root of numbers is equal to a squared root of their cube.
|
וכן שרש מרו' ממספרי' מושב באופן מעו' הכאה שוה ממה שעושה שרש אחד שוה בהיותו מעו' מוכה בעצמו באופן מרו' |
| Therefore, when the squares that are one part of the equation are cubed they become equal to the numbers that are the radicand of the cube root.
|
א"כ בהכות הצינסי אשר הם א' מהחלקי' מההשוא' באופן מעוק' עושה כמו המספרי' הנקובי' להיות להם שרש מעו' היותם עוד מספרי' |
| So [the equation] is returned to chapter 21: | א"כ בהשיבה אל הפרק הכ"א |
| That is, a root of a square of a square of a square i.e. a root of a cube of a cube becomes a cube and it is equal to a square root of a number, [whose cube root is cubed].
|
דהיינו אל שרש צינסי מצינסי מצינסי דהיינו שרש מעו' ממעו' יבא להיות מעו' ויהיה שוה אל שרש מרובע מהמספר מושב אל מעו' |
| Hence, a cube equals a root of a number.
|
א"כ מעו' יהיה שוה אל שרש מספר |
Chapter 50 |
פרק נ' |
| When numbers are equal to a cube root of squares:
|
כאשר המספרי' יהיו שוים אל שרשי' מעו' מצינסי |
| The numbers should be cubed. | צריך להשיב המספרי' אל מעו' |
| Then, the cubed number is divided by the number of the squares that are the radicand of the cube root. | ואותה ההשבה תחלק בכמות הצינסי הנקובי' להיות להם שרש מעו' |
| The square root of the result is equal to the thing.
|
ושרש מרוב' ממה שיבא מזה יבא לשוות הדבר |
|
והנה המשל עשה לי זה החשבון תמצא לי [55]שני מספרי' שיהיה הראשון חלק מהשני כמו שא' הוא מג' ומוכה שרש מעו' מהראשון בשרש מעו' השני יעשה ק' |
|
זאת היא פעולתו |
|
תניח שהמספר ראשון יהיה דבר אחד והשני ג' דברי' |
|
עתה תכה שרש המעו' מהראשון עם השרש מעו' מהשני שזהו שרש מעו' דבר אחד בשרש מעו' ג' דברי' ועולה שרש מעו' מג' צינסי והם שוים אל ק' |
|
עתה תרדוף כפי הכלל האמור למעלה |
|
תשיב מאה שהם המספרי' אל מעו' ויהיה לך אלף אלפים |
|
תחלקם בכמות הצינסי הנקובי' להיות להם שרש מעו' שזהו ג' ויבא מזה של"ג אלפי' ושל"ג ושליש |
|
ושרש המרו' מזה יבא לשוות הדבר ואתה הנחת היות המספר ראשון דבר אחד א"כ המספר ראשו' יבא להיות שרש משל"ג אלפי' ושל"ג ושליש |
|
והשני הנחת היותו ג' דברי' א"כ תכה ג' בשל"ג אלפי' ושל"ג ושליש ועולה שרש מג' אלפי אלפי' וככה יבא להיות המספר השני |
| Know that this equation can be returned to the second chapter, by converting the number to a cube. | ודע שזאת ההשואה אפשר להשיבה אל הפרק השני בהשיב המספר אל מעו' |
| "A cube [root] of a number is equal to a cube root of squares" is equal to your saying "the number is equal to squares".
|
יהיה מעו' מספר שוה אל שרש מעו' מצינסו ושוה לאמרך המספר שוה לצינסי |
Chapter 51 |
פרק נ"א |
| עוד רצוני לשומך באופן אחר | |
| It is when cubes are equal to a cube root of the numbers:
|
וזהו כאשר המעוקבי' יהיו שוים אל שרש מעו' ממספרי' |
| The number of the cubes should be cubed. | צריך להשיב כמות המעו' המספרי' אל מעו' |
| Then, the radicand of the cube root is divided by the cubed number of the cubes. | ולחלק כמות המספרי' הנקובי' להיות להם שרש מעו' בהשבת כמות המעו' אל מעו' |
| The cube root of the result is equal to the thing and its cube root is a [cube] root of its cube root.
|
ושרש המעו' ממה שיבא מזה ישוה הדבר ושרשו המעוק' הוא שרש משרשו המעו' |
|
והנה המשל תמצא לי שני מספרי' שיהיה אחד מהם חלק מהאחר כמו שא' הוא מד' ומוכה הראשון בשני וההכאה ההיא תוכה בראשון יעשה שרש מעו' מרי"ו |
|
זו היא פעלתו |
|
תניח שהמספר ראשון יהיה דבר אחד והאחר יהיה ד' דברי' |
|
עתה תכה הראשון בשני שזהו דבר אחד בד' דברי' עולה ד' צינסי |
|
וזאת ההכאה שהיא ד' צינסי תכה במספר ראשו' שהוא דבר אחד עולה ד' מעו' שהם שוים אל שרש מעו' מרי"ו |
|
עתה תרדוף כפי הכלל האמור למעלה |
|
הנך צריך להשיב כמות המעו' אל מעו' וזהו ד' ויהיה לך |
|
ותחלק כמות המספרי' הנקובי' להיות להם שרש מעו' והם רי"ו על ס"ד ויבא מזה ג' וג' מח' |
|
ושרש מעו' משרש מעו' מג' וג' מח' יבא להיות הדבר וככה יבא להיות המספר ראשון אשר הנחת היותו דבר אחד |
|
והשני הנחת היותו ד' דברי' א"כ תכה ד' בשרש מעו' משרש מעו' מג' וג' מח' |
|
ותזכור כי הנך צריך להשיב אל שרש מעו' משרש מעו' ויהיה לך שרש מעו' משרש מעו' מרס"ב אלפי' וקמ"ד |
|
וזה תכה בג' וג' מח' ועולה שרש מעו' משרש מעו' מתתפ"ד אלפי' ותשל"ו וככה יבא להיות המספ' השני[56] |
| Over and done | תם ונשלם |
| Praise to the Creator of the Universe | שבח לבורא עולם |
Notes
Appendix: Bibliography
Aliabraa Argibra / by Maestro Dardi (Pisa, 14th century)
– Hebrew translation –
by Mordecai (Angelo) Finzi (Mantua, d. 1475)
Jīblī al-Mūqabāla
Manuscripts:
- Jerusalem, The National Library of Israel Ms. Heb. 8°3915 (IMHM: B 546 (8°3915)), ff. 1r-43r (Mantova, 1473-1975; autograph)
- Paris, Bibliothèque Nationale de France heb. 1029/5 (IMHM: f 15721), ff. 194r-234r (15th-16th century)
- Paris, Bibliothèque Nationale de France heb. 1033/2 (IMHM: f 15026), ff. 91r-150v (16th century)
The transcript is based mainly on manuscript Jerusalem 8°3915
Critical Edition (of the first section):
- Wagner, Roy. 2013. Mordekhai Finzi's Translation of Maestro Dardi's Italian Algebra – a Partial Edition. In: Alexander Fidora, Harvey J. Hames, Yossef Schwarz eds. Latin-into-Hebrew Texts and Studies. Volume Two: Text in Contexts. Leiden, Boston: Brill, 2013, pp. 437-499.
Bibliography:
- Lévy, Tony. 2007. L’algèbre arabe dans les textes hébraïques (II). Dans l’Italie des XVe et XVIe siècles, sources arabes et sources vernaculaires, Arabic Sciences and Philosophy 17, pp. 81-107.
- Van Egmond, Warren. 1983. The Algebra of Maestro Dardi of Pisa, Historia Mathematica 10, pp. 399-421.
- Wagner, Roy. 2013. Mordekhai Finzi's Translation of Maestro Dardi's Italian Algebra. In: Alexander Fidora, Harvey J. Hames, Yossef Schwarz eds. Latin-into-Hebrew Texts and Studies. Volume Two: Text in Contexts. Leiden, Boston: Brill, 2013, pp. 195-221.
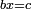
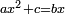
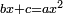
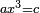
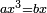
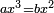
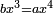
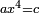
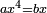
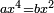
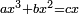
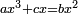
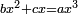
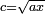
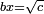
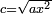
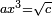


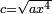
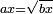
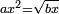
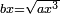
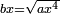
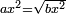

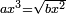
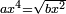
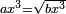
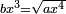
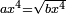
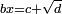
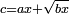
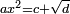

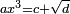
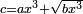
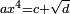
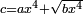
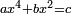

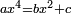
![\scriptstyle c=\sqrt[3]{bx}](/mediawiki/images/math/5/b/4/5b40a2184fb3c6324ff5fe2eedb9da0b.png)
![\scriptstyle ax^2=\sqrt[3]{c}](/mediawiki/images/math/f/c/0/fc0033e1c190540235d4afbc47b31b11.png)
![\scriptstyle c=\sqrt[3]{ax^2}](/mediawiki/images/math/f/7/6/f7617468fb35452327a7d2d5678e45f0.png)
![\scriptstyle ax^3=\sqrt[3]{c}](/mediawiki/images/math/a/6/4/a64e6eaeaf2d5f64610f741998d43d86.png)
![\scriptstyle c={\color{red}{\sqrt[3]{ax^3}}}](/mediawiki/images/math/7/d/0/7d0f000fa4e15b1c77284cac618571d0.png)
![\scriptstyle ax^4=\sqrt[3]{c}](/mediawiki/images/math/1/e/7/1e75351d5bb4c6692c0c764f8c41630c.png)
![\scriptstyle c=\sqrt[3]{ax^4}](/mediawiki/images/math/5/e/d/5ed81118b61c75402c62df045062825c.png)
![\scriptstyle ax=\sqrt[3]{bx}](/mediawiki/images/math/1/3/1/131226332966c099be04a0bd7b23bdb8.png)
![\scriptstyle bx=\sqrt[3]{ax^2}](/mediawiki/images/math/f/7/8/f78459344221b7260fa44eab44e97048.png)
![\scriptstyle ax^3=\sqrt[3]{bx}](/mediawiki/images/math/7/6/8/7687bc96d941e603e2226755d3d59c2b.png)
![\scriptstyle bx=\sqrt[3]{ax^4}](/mediawiki/images/math/d/6/5/d6531f1559e6a2635c1ec020e48ffa3c.png)
![\scriptstyle ax^2=\sqrt[3]{bx^2}](/mediawiki/images/math/5/8/8/58877d6e477060b7fae7a692e211e2e7.png)
![\scriptstyle ax^2=\sqrt[3]{bx^3}](/mediawiki/images/math/5/e/2/5e22d4f5e60f934e64b27aa681bd66c9.png)
![\scriptstyle ax^2=\sqrt[3]{bx^4}](/mediawiki/images/math/4/7/0/47054aef651f71b64777b681e7003bbc.png)
![\scriptstyle ax^3=\sqrt[3]{bx^3}](/mediawiki/images/math/c/4/f/c4f205465e9b0c3f3c6d96201bca75bb.png)
![\scriptstyle ax^4=\sqrt[3]{bx^3}](/mediawiki/images/math/a/8/f/a8f4e7c60e14d6b32152ff189c2c79ea.png)
![\scriptstyle ax^4=\sqrt[3]{bx^4}](/mediawiki/images/math/d/8/2/d8278732f6f39c68a3f585e57ee1d381.png)
![\scriptstyle ax=c+\sqrt[3]{d}](/mediawiki/images/math/2/4/0/240c5ecfde97586517c3bff2c0371ddd.png)
![\scriptstyle ax^2=c+\sqrt[3]{d}](/mediawiki/images/math/d/2/0/d20e2e16e9116c00de15fca3fa9ba7b6.png)
![\scriptstyle ax^3=c+\sqrt[3]{d}](/mediawiki/images/math/c/6/6/c6608d640c7379491e4543a1b2408848.png)
![\scriptstyle ax^4=c+\sqrt[3]{d}](/mediawiki/images/math/a/5/3/a53dd8319eae58d0432715c91c94ded9.png)
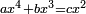
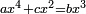

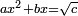
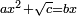
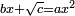
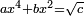

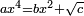
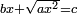
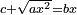


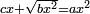

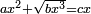

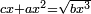
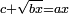

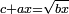
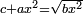
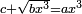
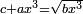
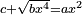
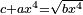
![\scriptstyle ax^2+bx=\sqrt[3]{c}](/mediawiki/images/math/a/d/6/ad6e0ba75374eb1808a175fc741ec668.png)
![\scriptstyle ax^2+\sqrt[3]{c}=bx](/mediawiki/images/math/4/f/c/4fca7691a8d3d65f06a9e0711f53c64a.png)
![\scriptstyle bx+\sqrt[3]{c}=ax^2](/mediawiki/images/math/5/6/4/56425d9f5144f7a115727e08200be0af.png)
![\scriptstyle ax^4+bx^2=\sqrt[3]{c}](/mediawiki/images/math/9/0/2/9024145570d9ac61bd0a30b9f4ab1ff3.png)
![\scriptstyle ax^4+\sqrt[3]{c}=bx^2](/mediawiki/images/math/7/0/f/70f5c392ff7f8a030d064abf63816a13.png)
![\scriptstyle ax^4=bx^2+\sqrt[3]{c}](/mediawiki/images/math/8/2/5/8258e76c3f17fd856dbd6704d5d93247.png)
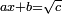
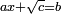

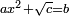
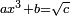
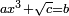
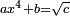
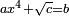
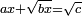
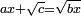
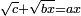
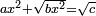
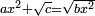
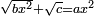
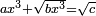
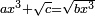
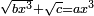

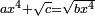
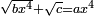
![\scriptstyle ax+\sqrt{bx}=\sqrt[3]{c}](/mediawiki/images/math/0/1/e/01e9d56074abfa66239fccf5285fa38f.png)
![\scriptstyle ax+\sqrt[3]{c}=\sqrt{bx}](/mediawiki/images/math/c/3/6/c36fb4ea4a608bd36697cf8a982e7587.png)
![\scriptstyle\sqrt{ax^2}+\sqrt[3]{c}=bx](/mediawiki/images/math/b/9/f/b9fb2f72c6a7fe66514779af121ffd61.png)
![\scriptstyle ax^2+\sqrt{bx^2}=\sqrt[3]{c}](/mediawiki/images/math/6/7/6/6764de3937205392c33439b6ea96e18c.png)
![\scriptstyle ax^2+\sqrt[3]{c}=\sqrt{bx^2}](/mediawiki/images/math/2/e/9/2e9b28ced07e6e19274f0984746c7a42.png)
![\scriptstyle\sqrt{bx^2}+\sqrt[3]{c}=ax^2](/mediawiki/images/math/d/1/4/d147ff02536cd92c44a35054b1ae4202.png)
![\scriptstyle ax^3+\sqrt{bx^3}=\sqrt[3]{c}](/mediawiki/images/math/a/4/6/a46113fd364f1a92f973831fe8d0417f.png)
![\scriptstyle ax^3+\sqrt[3]{c}={\color{red}{\sqrt{bx^3}}}](/mediawiki/images/math/9/2/5/925c350009d6ece26ca9e93a6265a45d.png)
![\scriptstyle\sqrt{bx^3}+\sqrt[3]{c}=ax^3](/mediawiki/images/math/b/a/1/ba1e392cb57a72c7cb306175f1974dbf.png)
![\scriptstyle ax^4+\sqrt{bx^4}=\sqrt[3]{c}](/mediawiki/images/math/3/8/0/380d40c56bf6e4f13cdf3042cecb2312.png)
![\scriptstyle ax^4+\sqrt[3]{c}=\sqrt{bx^4}](/mediawiki/images/math/d/b/2/db297403cdcb3efbec0574067c360e66.png)
![\scriptstyle\sqrt{bx^4}+\sqrt[3]{c}=ax^4](/mediawiki/images/math/9/2/8/9287b7dd0ee7db4b45ccc62b97a6661d.png)
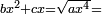
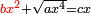
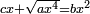
![\scriptstyle bx+d=\sqrt[3]{c}](/mediawiki/images/math/e/4/6/e4621fd35330c091a7903f45510c6ba0.png)
![\scriptstyle bx+\sqrt[3]{c}=d](/mediawiki/images/math/e/3/e/e3ebe4bd48fd1681f85e5b33abbe84bb.png)
![\scriptstyle {\color{red}{ax^2}}+d=\sqrt[3]{c}](/mediawiki/images/math/0/d/c/0dc11cbc1194178f7bb7ee13eeeea108.png)
![\scriptstyle ax^2+\sqrt[3]{c}=d](/mediawiki/images/math/6/d/a/6da68dcd63521931b1759ad05c4d172f.png)
![\scriptstyle ax^3+d=\sqrt[3]{c}](/mediawiki/images/math/7/2/8/728606a82983e02c0a10c59659d2f9b1.png)
![\scriptstyle ax^3+\sqrt[3]{c}=d](/mediawiki/images/math/6/8/8/688268580fd66487fa5d150f832e84b3.png)
![\scriptstyle ax^4+d=\sqrt[3]{c}](/mediawiki/images/math/1/0/1/10192372c4639861eed4d4f9514e55f6.png)
![\scriptstyle ax^4+\sqrt[3]{c}=d](/mediawiki/images/math/1/0/a/10ae0b5daf4067ed5227e959419b0503.png)


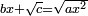
![\scriptstyle\sqrt{ax^2}+bx=\sqrt[3]{c}](/mediawiki/images/math/d/e/7/de74f9fdca2fe53dae255288f3d6d9ce.png)
![\scriptstyle bx+\sqrt[3]{c}=\sqrt{ax^2}](/mediawiki/images/math/f/4/e/f4ec3145cb8588d6c205a6fa94365ca7.png)
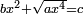
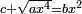
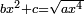

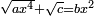

![\scriptstyle bx^2+\sqrt{ax^4}=\sqrt[3]{c}](/mediawiki/images/math/d/c/e/dce2fab1c6c5650da18e28f3e9d48990.png)
![\scriptstyle\sqrt{ax^4}+\sqrt[3]{c}=bx^2](/mediawiki/images/math/3/6/4/36487e744e7b3d3fffb09e03754a348e.png)
![\scriptstyle ax^2+\sqrt[3]{c}=\sqrt{bx^4}](/mediawiki/images/math/4/0/d/40d22a13eb4f246dc52d56afeb415e99.png)
![\scriptstyle\sqrt[3]{bx}=c](/mediawiki/images/math/2/f/0/2f0a49fd265b882a6d8ea9f5e92b1203.png)
![\scriptstyle\sqrt[3]{ax^2}=c](/mediawiki/images/math/a/6/5/a6500ae46f38192403c6cd7e0380f6b1.png)
![\scriptstyle\sqrt[3]{ax^3}=c](/mediawiki/images/math/6/c/6/6c6dc053745fc53a948ae71e3f5b9062.png)
![\scriptstyle\sqrt[3]{ax^4}=c](/mediawiki/images/math/8/3/5/835c93b537d54579ad4e7efc3d7baef6.png)
![\scriptstyle\sqrt[3]{bx}=\sqrt{c}](/mediawiki/images/math/5/c/a/5caf482181d34c7ebf0d593ca900d298.png)
![\scriptstyle\sqrt[3]{ax^2}=\sqrt{c}](/mediawiki/images/math/6/1/3/6131aa6c46fc8ca4f9451afe499cda0b.png)
![\scriptstyle\sqrt[3]{ax^3}=\sqrt{c}](/mediawiki/images/math/1/0/7/10715442ffd6877b77529bce3b31ecc4.png)
![\scriptstyle\sqrt[3]{ax^4}=\sqrt{c}](/mediawiki/images/math/2/a/0/2a02b522cccac89301514914005a142b.png)
![\scriptstyle\sqrt{bx}=\sqrt[3]{c}](/mediawiki/images/math/9/9/b/99b430b5938a143790b7e259de525f98.png)
![\scriptstyle\sqrt{ax^2}=\sqrt[3]{c}](/mediawiki/images/math/a/6/4/a642a8ad92449b522f85bc1ace17fdca.png)
![\scriptstyle\sqrt{ax^3}=\sqrt[3]{c}](/mediawiki/images/math/4/7/7/4772e0c3a0cb77d744fd370b10ed6ef9.png)
![\scriptstyle\sqrt{ax^4}=\sqrt[3]{c}](/mediawiki/images/math/0/f/d/0fd3d94216686464ff65cedf3c022d06.png)
![\scriptstyle\sqrt{ax}=\sqrt[3]{bx}](/mediawiki/images/math/c/f/9/cf97cef919ef38813dc50fef5de47a6b.png)
![\scriptstyle\sqrt{ax^2}=\sqrt[3]{bx^2}](/mediawiki/images/math/e/8/b/e8bf488dfcb249b1615cc6750864a2de.png)
![\scriptstyle\sqrt{ax^3}=\sqrt[3]{bx^3}](/mediawiki/images/math/4/1/b/41b24da887d2cdee3690c7eb34f4b5a8.png)
![\scriptstyle\sqrt{ax^4}=\sqrt[3]{bx^4}](/mediawiki/images/math/9/d/3/9d38fd4ee516eb180d24f7f34c8bd75c.png)
![\scriptstyle\sqrt{bx}=\sqrt[3]{ax^2}](/mediawiki/images/math/c/b/d/cbd7e22686ad9ba49d8ef61dbfa21583.png)
![\scriptstyle\sqrt{bx}=\sqrt[3]{ax^3}](/mediawiki/images/math/2/f/a/2facf00396fcc963b935ce306c39d31f.png)
![\scriptstyle\sqrt{ax}=\sqrt[3]{bx^4}](/mediawiki/images/math/8/8/2/8827a98ac726d6ff7420b83e5891a9a7.png)
![\scriptstyle\sqrt{ax^2}=\sqrt[3]{bx}](/mediawiki/images/math/1/3/8/138540a0099c0b15231cde290183ede9.png)
![\scriptstyle\sqrt{ax^3}=\sqrt[3]{bx}](/mediawiki/images/math/c/5/c/c5cd92fc02b5cfe29e61591e3e989207.png)
![\scriptstyle\sqrt{ax^4}=\sqrt[3]{bx^2}](/mediawiki/images/math/e/f/f/eff46afae0af79770d472003408f226e.png)
![\scriptstyle\sqrt{ax^3}=\sqrt[3]{bx^4}](/mediawiki/images/math/8/8/2/882fd4fb7454f14b9aeb8717f8d0b9a5.png)
![\scriptstyle\sqrt{ax^4}=\sqrt[3]{bx^3}](/mediawiki/images/math/f/6/6/f66a56bf2e4b253c7e3896ffda79152b.png)
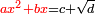
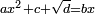
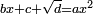
![\scriptstyle ax^2+bx=c+\sqrt[3]{d}](/mediawiki/images/math/d/7/9/d799841057f93bcdb531e1c83f489386.png)
![\scriptstyle ax^2+c+\sqrt[3]{d}=bx](/mediawiki/images/math/b/b/9/bb90fc410e8a537736b4baba3f66e46a.png)
![\scriptstyle bx+c+\sqrt[3]{d}=ax^2](/mediawiki/images/math/3/a/b/3ab9cbc37ed68d6208db8c9d9a2f3a32.png)
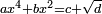
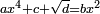
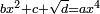
![\scriptstyle ax^4+bx^2=c+\sqrt[3]{d}](/mediawiki/images/math/6/a/c/6ac223aabcf9868c9c58cdaa565c167f.png)
![\scriptstyle ax^4+c+\sqrt[3]{d}=bx^2](/mediawiki/images/math/8/b/e/8be3907ce7fe7db8f931a45a9bd9ae21.png)
![\scriptstyle bx^2+c+\sqrt[3]{d}=ax^4](/mediawiki/images/math/f/7/c/f7cb79fa1dd05ac29aab97979683bcaa.png)

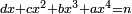
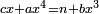
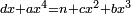
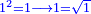


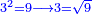
![\scriptstyle{\color{blue}{1^3=1^2\sdot1=1\sdot1=1\longrightarrow1=\sqrt[3]{1}}}](/mediawiki/images/math/6/5/8/658c52eaa68629c3199dd21f49dcc38d.png)
![\scriptstyle{\color{blue}{2^2\sdot2=4\sdot2=8\longrightarrow2=\sqrt[3]{8}}}](/mediawiki/images/math/9/b/a/9ba74d157b8046c7fa13f6ad696b4a21.png)
![\scriptstyle{\color{blue}{3^2\sdot3=9\sdot3=27\longrightarrow3=\sqrt[3]{27}}}](/mediawiki/images/math/f/2/5/f25ebe1e29b6dfc5e66e817925e63d33.png)
![\scriptstyle{\color{blue}{1=\sqrt{1}=\sqrt[3]{1}}}](/mediawiki/images/math/a/6/6/a6617fc4b659029dedf699f630c3af46.png)
![\scriptstyle{\color{blue}{2=\sqrt{4}=\sqrt[3]{8}}}](/mediawiki/images/math/6/7/a/67a51de5e59e529a528b61c52c1b317d.png)
![\scriptstyle{\color{blue}{3=\sqrt{9}=\sqrt[3]{27}}}](/mediawiki/images/math/9/a/5/9a5f5712a95a5fea25c6a51482d81dfd.png)
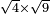
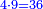
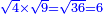
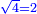 ;
; 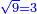




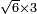
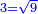


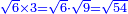
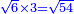
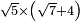




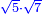
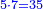
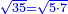
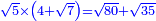
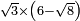

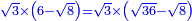
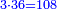

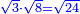
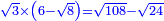


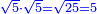
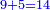


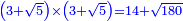
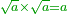
![\scriptstyle{\color{OliveGreen}{\left(a+\sqrt{b}\right)\times\left(a+\sqrt{b}\right)=\left[a\sdot\left(a+\sqrt{b}\right)\right]+b=\left(a\sdot a\right)+\sqrt{\left(a+a\right)^2\sdot b}+b}}](/mediawiki/images/math/b/f/5/bf5df0270ea88fed99fe7f59d3a9f13f.png)

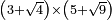
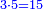

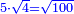
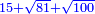
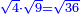
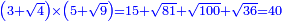
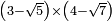
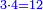

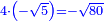
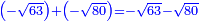


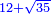
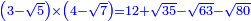

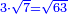
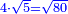
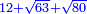
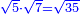
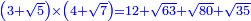
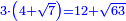

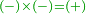
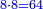

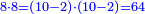
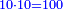

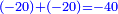


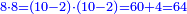
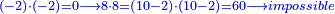

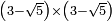
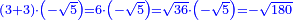
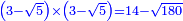
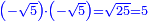
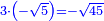


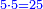
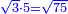
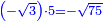

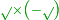
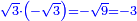
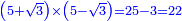
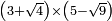


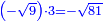
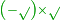
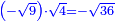
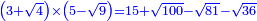
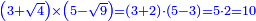
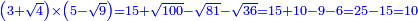
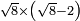
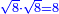
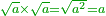
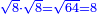
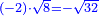
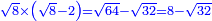
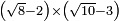

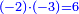


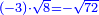
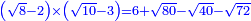
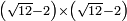
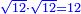
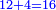
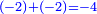
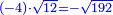
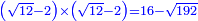
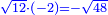
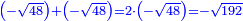
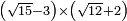

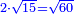
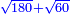
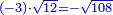

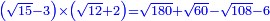
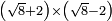
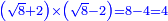
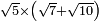

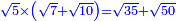
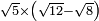

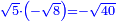
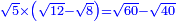
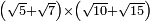



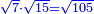
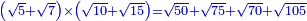
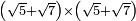
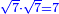
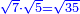
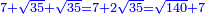
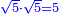
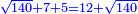
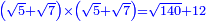
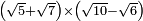

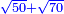

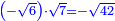
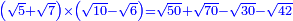

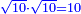
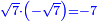
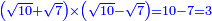
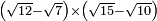





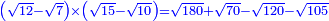
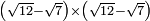
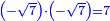

![\scriptstyle{\color{blue}{\left[\sqrt{12}\sdot\left(-\sqrt{7}\right)\right]+\left[\left(-\sqrt{7}\right)\sdot\sqrt{12}\right]=2\sdot\left({\color{red}{-}}\sqrt{84}\right)=-\sqrt{336}}}](/mediawiki/images/math/3/3/b/33ba1f66815d2ec2bc616179474fe3d5.png)

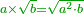
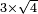
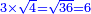
![\scriptstyle3\times\sqrt[3]{8}](/mediawiki/images/math/1/3/2/1328cc433a8d6f068d82010e6cd86a87.png)
![\scriptstyle{\color{blue}{3=\sqrt[3]{27}}}](/mediawiki/images/math/2/2/0/22009970c5809c09c1756dc0f0d61e2a.png)
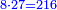
![\scriptstyle{\color{blue}{3\times\sqrt[3]{8}=\sqrt[3]{216}=6}}](/mediawiki/images/math/2/9/4/29450a0652084fe2c397e6ae73d8e551.png)
![\scriptstyle\sqrt{4}\times\sqrt[3]{8}](/mediawiki/images/math/6/4/6/646c5ba2e5f167061f7a7ccea09fdf58.png)
![\scriptstyle{\color{blue}{4=\sqrt[3]{64}}}](/mediawiki/images/math/a/8/5/a85927497f2680d740a83df67fdeb40a.png)

![\scriptstyle{\color{blue}{\sqrt{\sqrt[3]{64}}\sdot\sqrt{\sqrt[3]{64}}=\sqrt{\sqrt[3]{64}}\times\sqrt[3]{\sqrt{64}}}}](/mediawiki/images/math/8/c/d/8cd7291c0e6c76c514bfb115c3f92036.png)
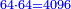
![\scriptstyle{\color{blue}{\sqrt{4}\times\sqrt[3]{8}=\sqrt{\sqrt[3]{4096}}=\sqrt[3]{\sqrt{4096}}=4}}](/mediawiki/images/math/e/c/9/ec96c4973e1f53ba45e20d1e5f3bab09.png)
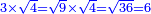
![\scriptstyle{\color{blue}{3\times\sqrt[3]{8}=\sqrt[3]{27}\times\sqrt[3]{8}=\sqrt[3]{216}=6}}](/mediawiki/images/math/a/9/c/a9ccaf914642eeb30466f3d21c094576.png)
![\scriptstyle{\color{blue}{\sqrt{4}\times\sqrt[3]{8}=\sqrt{\sqrt[3]{4096}}}}](/mediawiki/images/math/1/1/4/11434a961de6331559f9667757032b88.png)
![\scriptstyle\sqrt[3]{8}\times\sqrt[4]{16}](/mediawiki/images/math/b/e/2/be2421d68cb602563f762c6471b4910a.png)


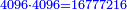
![\scriptstyle{\color{blue}{\sqrt[3]{8}\times\sqrt[4]{16}=\sqrt[3]{\sqrt[4]{16777216}}=\sqrt[4]{\sqrt[3]{16777216}}=4}}](/mediawiki/images/math/c/c/3/cc37c387b3235346b445d7f7c4a81181.png)
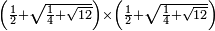
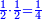

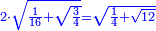
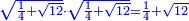
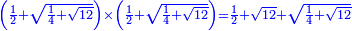
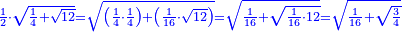
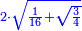
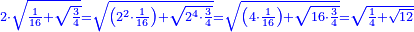


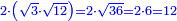
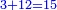


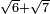
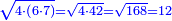

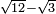

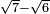
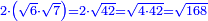
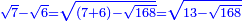
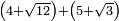
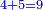
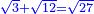

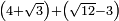

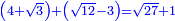
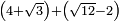
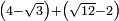

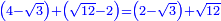
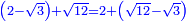

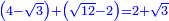
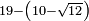
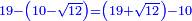
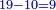
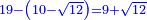
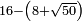


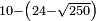
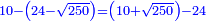
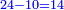

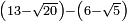


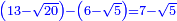
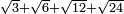
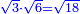


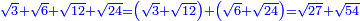

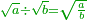
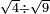
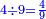
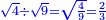
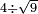
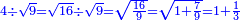
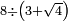
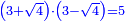
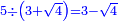
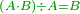
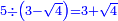
![\scriptstyle{\color{blue}{\left[5\div\left(3+\sqrt{4}\right)\right]:\left(3-\sqrt{4}\right)=\left[8\div\left(3+\sqrt{4}\right)\right]:a}}](/mediawiki/images/math/a/8/3/a83780ae9aae5fb7984297197f60532c.png)


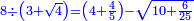
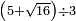

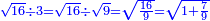
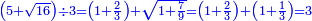
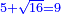
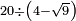
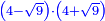 = 7
= 7
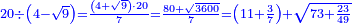
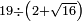
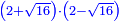

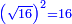
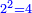


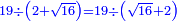
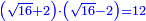
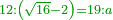
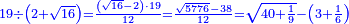
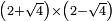
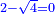
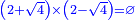
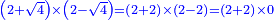
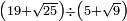
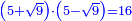

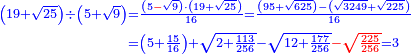
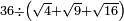
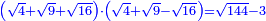
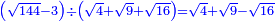
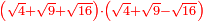 =
= 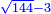
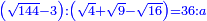

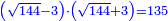

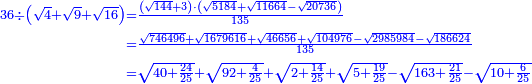


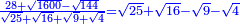


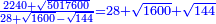
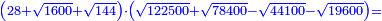
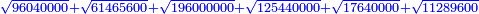


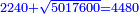


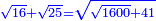
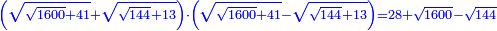
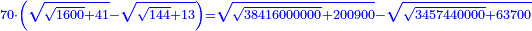





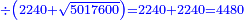

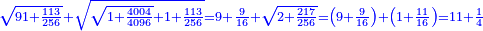

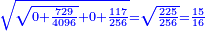
![\scriptstyle{\color{blue}{70\div\left(\sqrt{4}+\sqrt{9}+\sqrt{16}+\sqrt{25}\right)=\left(11+\frac{1}{4}\right)-\left[\left(5+\frac{5}{16}\right)+\frac{15}{16}\right]=\left(11+\frac{1}{4}\right)-\left(6+\frac{1}{4}\right)=5}}](/mediawiki/images/math/8/0/f/80f279d12ebe068761143cf1a1d81606.png)
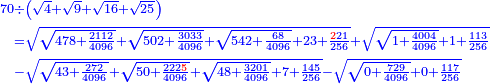

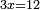
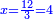

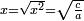
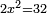
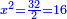

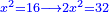
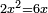
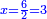


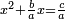

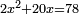
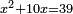
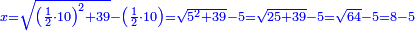
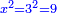
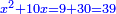
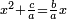
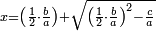
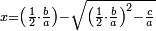

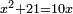
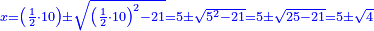
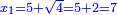
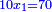
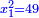
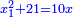
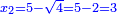

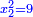
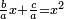
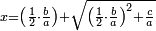
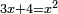
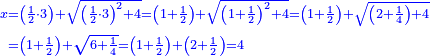
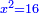
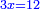
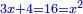

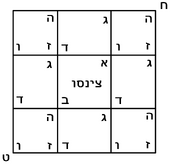
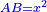
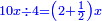

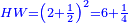
![\scriptstyle{\color{blue}{\left[AB+4GD\right]=x^2+10x}}](/mediawiki/images/math/d/7/e/d7e4ae28472caf4782d1d9c82cc1b6e8.png)
![\scriptstyle{\color{blue}{\left[4HW\right]=25}}](/mediawiki/images/math/7/8/e/78e3a4eb0cb77fa26804c69cfc25691e.png)
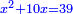

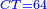
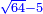 ].
].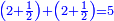
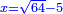
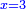

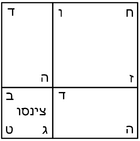
 ] and write BG on this surface.
] and write BG on this surface.


![\scriptstyle{\color{blue}{\left[BG+2DH\right]=x^2+10x=39}}](/mediawiki/images/math/1/4/c/14cc2169c2795774ddf458b9d6e63a73.png)
![\scriptstyle{\color{blue}{\left[BG+2DH\right]+WZ=39+25=64}}](/mediawiki/images/math/5/5/6/5564289131a01e53ca29eae606144ae2.png)
![\scriptstyle{\color{blue}{\left[BG+2DH+WZ\right]=CT=64}}](/mediawiki/images/math/0/d/c/0dc03df4a5c8706a7e4834af563c660a.png)
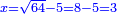
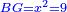
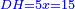

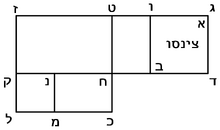
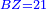
![\scriptstyle{\color{blue}{\left[DZ\right]=10x}}](/mediawiki/images/math/b/4/2/b42c2eb6e8a21e7e42e9fd783f8ebb99.png)

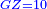
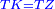
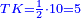


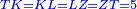
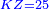

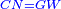
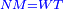
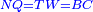
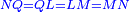

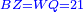
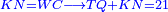

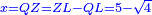
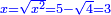
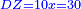
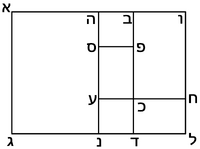
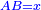

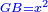
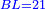

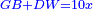
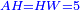

![\scriptstyle{\color{blue}{\left[EC\right]\parallel WH}}](/mediawiki/images/math/3/8/3/38336711705746729fb73f92acf883af.png)
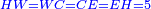
![\scriptstyle{\color{blue}{\left[EW\right]=25}}](/mediawiki/images/math/0/d/8/0d8a5d051faac38275bcb921f17adc57.png)
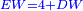
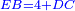
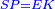
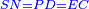
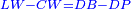
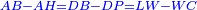
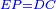



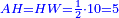
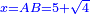
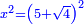
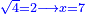
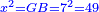
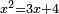


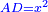
![\scriptstyle{\color{blue}{\left[BP\right]\parallel AH}}](/mediawiki/images/math/8/5/f/85f4d3585127f5158e7a9b3cc7968039.png)

![\scriptstyle{\color{blue}{\left[HB+HD\right]=4+3x=x^2=\left[AD\right]\longrightarrow HB=4}}](/mediawiki/images/math/3/1/0/3105098eac1dba905963d4e3bb549f79.png)
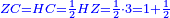
![\scriptstyle{\color{blue}{\left[HK\right]\parallel\left[CT\right]\quad HK=CT=HC=1+\frac{1}{2}}}](/mediawiki/images/math/c/7/0/c7044a476334667f061dd45247564e73.png)

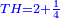

![\scriptstyle{\color{blue}{\left[LM\right]\parallel CA}}](/mediawiki/images/math/a/5/d/a5d84037b97612180a357d3480d114d2.png)
![\scriptstyle{\color{blue}{\left[LM\right]=CA=CL=AM}}](/mediawiki/images/math/1/9/c/19c74e2208c4392844daeecdba92dc68.png)
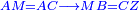
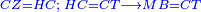




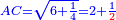
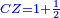


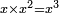
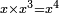
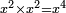
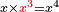
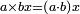

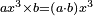
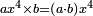

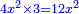

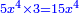


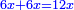
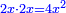
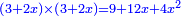
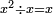
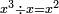
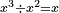
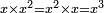
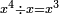

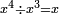
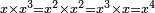



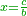
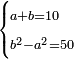
 ].
].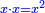
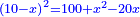
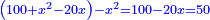

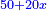


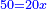

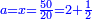
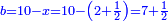
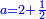
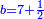
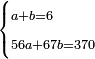
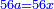 dinar
dinar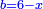 cubits
cubits dinar
dinar

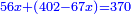

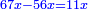
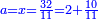

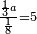

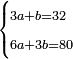
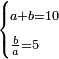
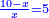

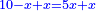
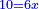
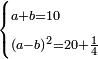


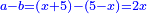
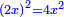
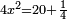
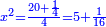
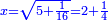
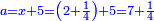
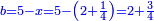

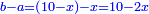
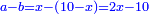
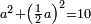
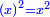
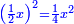
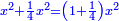
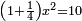
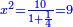
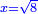
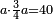

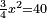
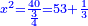
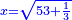
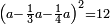

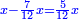
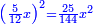
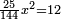
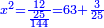
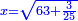
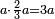

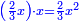
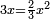
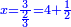
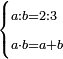
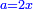
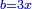

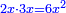

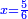

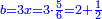
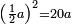
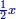
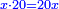

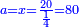
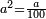
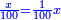
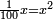
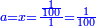
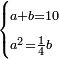
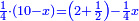
![\scriptstyle{\color{blue}{\left[\left(2+\frac{1}{2}\right)-\frac{1}{4}x\right]^2=\left(6+\frac{1}{4}\right)+\frac{1}{16}x^2-\left(1+\frac{1}{4}\right)x}}](/mediawiki/images/math/a/6/c/a6c27d26724511564d09571fe3f8e199.png)
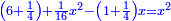
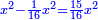

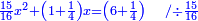
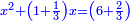
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle a=x&\scriptstyle=\sqrt{\left[\frac{1}{2}\sdot\left(1+\frac{1}{3}\right)\right]^2+\left(6+\frac{2}{3}\right)}-\left[\frac{1}{2}\sdot\left(1+\frac{1}{3}\right)\right]=\sqrt{\left(\frac{2}{3}\right)^2+\left(6+\frac{2}{3}\right)}-\frac{2}{3}\\&\scriptstyle=\sqrt{\frac{4}{9}+\left(6+\frac{2}{3}\right)}-\frac{2}{3}=\sqrt{7+\frac{1}{9}}-\frac{2}{3}\\\end{align}}}](/mediawiki/images/math/6/d/1/6d181f297590540b74e72ded6841412f.png)
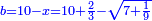
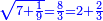
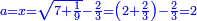

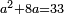
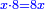
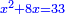
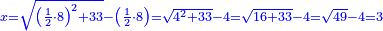
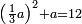
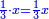
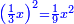
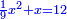
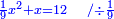
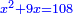
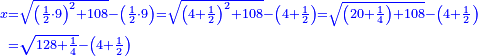


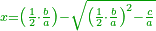
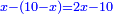
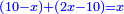
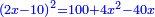
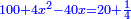
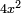 :
: 
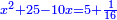
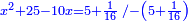
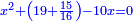
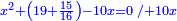

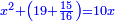

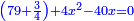




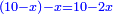

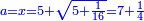
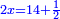
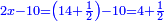
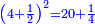
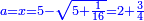
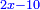 impossible
impossible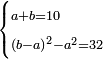

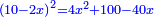

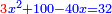
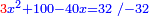



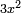 :
: 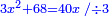
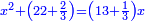
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle a=x&\scriptstyle=\left[\frac{1}{2}\sdot\left(13+\frac{1}{3}\right)\right]-\sqrt{\left[\frac{1}{2}\sdot\left(13+\frac{1}{3}\right)\right]^2-\left(22+\frac{2}{3}\right)}\\&\scriptstyle=\left(6+\frac{2}{3}\right)-\sqrt{\left(6+\frac{2}{3}\right)^2-\left(22+\frac{2}{3}\right)}\\&\scriptstyle=\left(6+\frac{2}{3}\right)-\sqrt{\left(44+\frac{4}{9}\right)-\left(22+\frac{2}{3}\right)}\\&\scriptstyle=\left(6+\frac{2}{3}\right)-\sqrt{21+\frac{7}{9}}\\\end{align}}}](/mediawiki/images/math/1/5/c/15c3120c2677f7db52afd36d85e9cdb4.png)
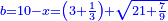
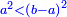
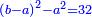
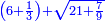 greater than the sum of the two parts, which should be ten
greater than the sum of the two parts, which should be ten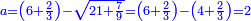
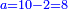

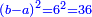
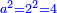
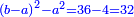
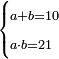
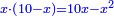

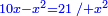

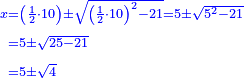
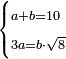
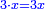
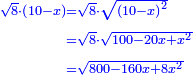
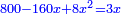


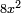 :
: 
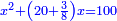
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle x&\scriptstyle=\left[\frac{1}{2}\sdot\left(20+\frac{3}{8}\right)\right]+\sqrt{\left[\frac{1}{2}\sdot\left(20+\frac{3}{8}\right)\right]^2-100}=\left(10+\frac{3}{16}\right)+\sqrt{\left(10+\frac{3}{16}\right)^2-100}\\&\scriptstyle=\left(10+\frac{3}{16}\right)+\sqrt{\left(103+\frac{201}{256}\right)-100}\\&\scriptstyle=\left(10+\frac{3}{16}\right)+\sqrt{3+\frac{201}{256}}\\\end{align}}}](/mediawiki/images/math/9/b/a/9ba50ae8c7bd695d2d11e238b6e37aec.png)




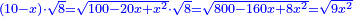
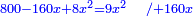
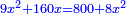
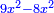

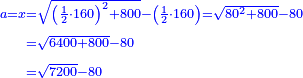
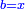
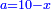

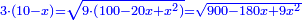
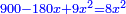
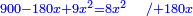
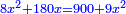
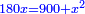

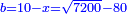
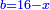



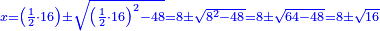
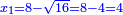



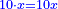
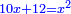

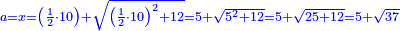


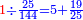
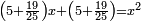
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle a=x&\scriptstyle=\left[\frac{1}{2}\sdot\left(5+\frac{19}{25}\right)\right]+\sqrt{\left[\frac{1}{2}\sdot\left(5+\frac{19}{25}\right)\right]^2+\left(5+\frac{19}{25}\right)}=\left(2+\frac{22}{25}\right)+\sqrt{\left(2+\frac{22}{25}\right)^2+\left(5+\frac{19}{25}\right)}\\&\scriptstyle=\left(2+\frac{22}{25}\right)+\sqrt{\left(8+\frac{184}{625}\right)+\left(5+\frac{19}{25}\right)}=\left(2+\frac{22}{25}\right)+\sqrt{14+\frac{34}{625}}\\\end{align}}}](/mediawiki/images/math/4/d/3/4d36a8c882655d3a16cafa48f5473065.png)
![\scriptstyle{\color{OliveGreen}{x=\sqrt[3]{\frac{c}{a}}}}](/mediawiki/images/math/4/e/1/4e167fdfcd9b12fc054bc49840549f76.png)
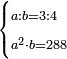
 ] and the other is four things [
] and the other is four things [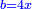 ]
]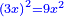
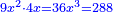
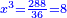
![\scriptstyle{\color{blue}{x=\sqrt[3]{x^3}=\sqrt[3]{8}=2}}](/mediawiki/images/math/f/f/f/fff7bced794923264ce2361aa2128271.png)
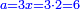
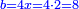
![\scriptstyle{\color{blue}{a=3x=3\sdot\sqrt[3]{8}=\sqrt[3]{27}\sdot\sqrt[3]{8}=\sqrt[3]{216}=6}}](/mediawiki/images/math/3/8/0/380d388384f9b68e7353dc5874828ff1.png)
![\scriptstyle{\color{blue}{b=4x=4\sdot\sqrt[3]{8}=\sqrt[3]{64}\sdot\sqrt[3]{8}=\sqrt[3]{512}=8}}](/mediawiki/images/math/5/c/1/5c1b9d40d765a9fd33a08168147e4acb.png)

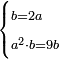
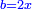 ], which is its double.
], which is its double.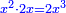

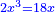
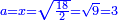
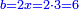
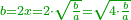
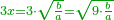
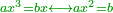
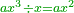
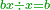
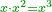
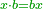
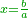
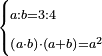

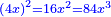
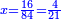

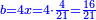
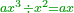
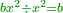
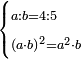
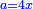 ] and the second is five things [
] and the second is five things [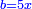 ].
].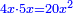
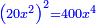
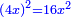
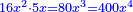



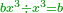
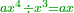
![\scriptstyle{\color{OliveGreen}{x=\sqrt[4]{\frac{c}{a}}}}](/mediawiki/images/math/d/e/3/de328ae1fa48e8542081bed3f56d0d76.png)
![\scriptstyle\left[a\sdot\left(\frac{2}{3}\sdot a\right)\right]^2=36](/mediawiki/images/math/4/4/d/44d26474e128ec5582fe301dd2b9863a.png)
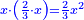
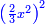
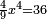
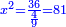
![\scriptstyle{\color{blue}{a=x=\sqrt[4]{81}=3}}](/mediawiki/images/math/e/a/a/eaaee42e5062e5b0ae3bc5445f4461dd.png)
![\scriptstyle{\color{OliveGreen}{x=\sqrt[3]{\frac{b}{a}}}}](/mediawiki/images/math/3/e/6/3e62942a07c03f87758993230147129d.png)
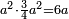
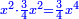


![\scriptstyle{\color{blue}{x=\sqrt[3]{\frac{6}{\frac{3}{4}}}=\sqrt[3]{8}=2}}](/mediawiki/images/math/e/f/9/ef9a6c4aa6ca64600c280f6a672a2fe9.png)




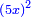
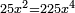
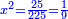
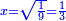

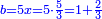
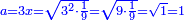

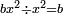
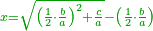
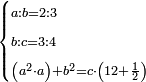
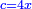
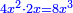
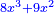
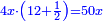
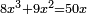
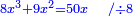
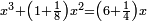
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle x&\scriptstyle=\sqrt{\left[\frac{1}{2}\sdot\left(1+\frac{1}{8}\right)\right]^2+\left(6+\frac{1}{4}\right)}-\left[\frac{1}{2}\sdot\left(1+\frac{1}{8}\right)\right]=\sqrt{\left(\frac{9}{16}\right)^2+\left(6+\frac{1}{4}\right)}-\frac{9}{16}=\sqrt{\frac{81}{256}+\left(6+\frac{1}{4}\right)}-\frac{9}{16}\\&\scriptstyle=\sqrt{6+\frac{145}{256}}-\frac{9}{16}=\left(2+\frac{9}{16}\right)-\frac{9}{16}=2\\\end{align}}}](/mediawiki/images/math/c/e/2/ce20da7fe6f326f0ff3ad904d3b8808a.png)



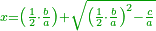

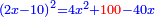

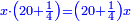

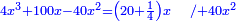
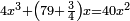
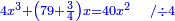
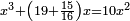
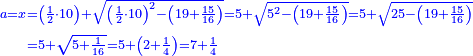
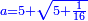
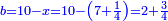


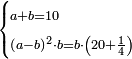


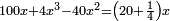
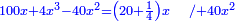
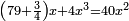

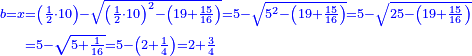
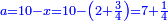
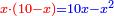
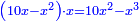

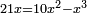
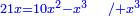
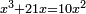
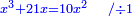




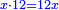
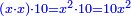
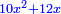
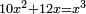


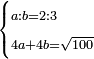
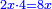
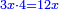
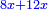
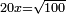
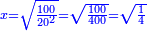
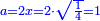
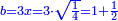
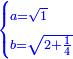

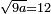

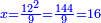
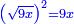
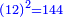
![\scriptstyle{\color{OliveGreen}{x=\sqrt[4]{\frac{c}{a^2}}}}](/mediawiki/images/math/d/c/6/dc6cfe5cc154ed3643087b6d773262a9.png)
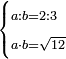
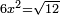
![\scriptstyle{\color{blue}{x=\sqrt[4]{\frac{12}{6^2}}=\sqrt[4]{\frac{12}{36}}=\sqrt[4]{\frac{1}{3}}}}](/mediawiki/images/math/8/d/b/8dbd35e8e9c020191d0e0ff1ce6f9306.png)
![\scriptstyle{\color{blue}{a=2x=2\sdot\sqrt[4]{\frac{1}{3}}=\sqrt[4]{5+\frac{1}{3}}}}](/mediawiki/images/math/1/7/7/177c891c69b1a9f4d271964c25263649.png)
![\scriptstyle{\color{blue}{b=3x=3\sdot\sqrt[4]{\frac{1}{3}}=\sqrt[4]{27}}}](/mediawiki/images/math/b/1/8/b18777e522a6f0c244f193ba832d7bc2.png)
 into roots
into roots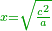

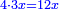

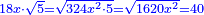
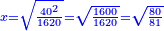
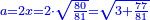

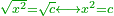
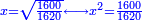


![\scriptstyle{\color{OliveGreen}{x=\sqrt[6]{\frac{c}{a^2}}}}](/mediawiki/images/math/c/9/c/c9c27e26579aef3a7251203b0b50c91c.png)

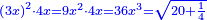
![\scriptstyle{\color{blue}{x=\sqrt[6]{\frac{20+\frac{1}{4}}{36^2}}=\sqrt[6]{\frac{20+\frac{1}{4}}{1296}}=\sqrt[6]{\frac{1}{64}}}}](/mediawiki/images/math/d/2/8/d280658b5c1563d5f27d1f5186ec0703.png)
![\scriptstyle{\color{blue}{a=3x=3\sdot\sqrt[6]{\frac{1}{64}}=\sqrt[6]{\frac{729}{64}}=1+\frac{1}{2}}}](/mediawiki/images/math/3/f/6/3f6acb3785d265fd9ebf56016841f668.png)
![\scriptstyle{\color{blue}{b=4x=4\sdot\sqrt[6]{\frac{1}{64}}=\sqrt[6]{\frac{4{\color{red}{0}}96}{64}}=2}}](/mediawiki/images/math/5/c/c/5cca5aa774a3e29f45e6283fd11e14da.png)
![\scriptstyle{\color{OliveGreen}{x=\sqrt[3]{\frac{c^2}{a}}}}](/mediawiki/images/math/2/b/a/2ba02df4134a10032cf434a5a2dd0ece.png)
![\scriptstyle\begin{cases}\scriptstyle a:b=3:5\\\scriptstyle\left[\left(a\sdot\sqrt{a}\right)+\left(b\sdot\sqrt{b}\right)\right]\sdot\sqrt{8}=100\end{cases}](/mediawiki/images/math/7/1/1/71187e61ee927d7bba48ce232ff213cc.png)
 ] is inexpressible, the first number should have been defined as three squares [
] is inexpressible, the first number should have been defined as three squares [ ], and the second number as five squares
], and the second number as five squares  , as the square has a proper root, which is one thing [
, as the square has a proper root, which is one thing [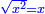 ].
]. ] is similar to the product of 3 squares by their root [
] is similar to the product of 3 squares by their root [ ], which is as many things as a root of 3.
], which is as many things as a root of 3.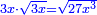
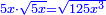
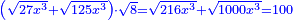
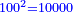
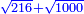
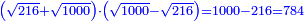
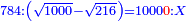
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle x&\scriptstyle=\sqrt[3]{\frac{1000{\color{red}{0}}\sdot\left(\sqrt{1000}-\sqrt{216}\right)}{784}}=\sqrt[3]{\frac{\sqrt{100000000{\color{red}{000}}}-\sqrt{216000000{\color{red}{00}}}}{784}}\\&\scriptstyle=\sqrt[3]{\sqrt{1626{\color{red}{00}}+\frac{569344{\color{red}{00}}}{614656}}-\sqrt{351{\color{red}{00}}+\frac{255744{\color{red}{00}}}{614656}}}\\\end{align}}}](/mediawiki/images/math/c/7/f/c7fb233c4a2c4ae8dca4fd8af0b7c34e.png)
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle a=3x&\scriptstyle=3\sdot\sqrt[3]{\sqrt{1626{\color{red}{00}}+\frac{569344{\color{red}{00}}}{614656}}-\sqrt{351{\color{red}{00}}+\frac{255744{\color{red}{00}}}{614656}}}\\&\scriptstyle=\sqrt[3]{\sqrt{729}}\sdot\sqrt[3]{\sqrt{1626{\color{red}{00}}+\frac{569344{\color{red}{00}}}{614656}}-\sqrt{351{\color{red}{00}}+\frac{255744{\color{red}{00}}}{614656}}}\\&\scriptstyle=\sqrt[3]{\sqrt{1186029{\color{red}{00}}+\frac{158976{\color{red}{00}}}{614656}}-\sqrt{256182{\color{red}{00}}+\frac{196608{\color{red}{00}}}{614656}}}\\\end{align}}}](/mediawiki/images/math/5/6/c/56c18492a4c62f4785c257fa1fd3d8f5.png)
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle b=5x&\scriptstyle=5\sdot\sqrt[3]{\sqrt{1626{\color{red}{00}}+\frac{569344{\color{red}{00}}}{614656}}-\sqrt{351{\color{red}{00}}+\frac{255744{\color{red}{00}}}{614656}}}\\&\scriptstyle=\sqrt[3]{\sqrt{15625}}\sdot\sqrt[3]{\sqrt{1626{\color{red}{00}}+\frac{569344{\color{red}{00}}}{614656}}-\sqrt{351{\color{red}{00}}+\frac{255744{\color{red}{00}}}{614656}}}\\&\scriptstyle=\sqrt[3]{\sqrt{25420723{\color{red}{00}}+\frac{83712{\color{red}{00}}}{614656}}-\sqrt{5490876{\color{red}{00}}+\frac{121344{\color{red}{00}}}{614656}}}\\\end{align}}}](/mediawiki/images/math/d/d/f/ddfa1b1def56ea4bb17c28ee2bb1a04d.png)
![\scriptstyle{\color{OliveGreen}{x=\sqrt[8]{\frac{c}{a^2}}}}](/mediawiki/images/math/b/e/1/be1f778de365af79dec554775005282a.png)
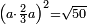
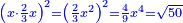
![\scriptstyle{\color{blue}{x=\sqrt[8]{\frac{50}{\left(\frac{4}{9}\right)^2}}=\sqrt[8]{\frac{50}{\frac{16}{81}}}=\sqrt[8]{253+\frac{1}{8}}}}](/mediawiki/images/math/a/9/7/a9791024550b08e8ee68e84fb760b997.png)
![\scriptstyle{\color{OliveGreen}{x=\sqrt[4]{\frac{c^2}{a}}}}](/mediawiki/images/math/c/2/8/c28d7c5cbdd9e3ece4084654ac46162a.png)
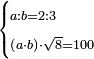
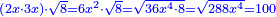
![\scriptstyle{\color{blue}{x=\sqrt[4]{\frac{100^2}{288}}=\sqrt[4]{\frac{10000}{288}}=\sqrt[4]{34+\frac{13}{18}}}}](/mediawiki/images/math/4/f/0/4f0db482d6733fc43a97bd2e268551fb.png)
![\scriptstyle{\color{blue}{a=2x=2\sdot\sqrt[4]{34+\frac{13}{18}}=\sqrt[4]{16\sdot\left(34+\frac{13}{18}\right)}=\sqrt[4]{555+{\color{red}{\frac{10}{18}}}}}}](/mediawiki/images/math/d/8/8/d88c496f84b948ea9bd81b6419f1ba8e.png)
![\scriptstyle{\color{blue}{b=3x=3\sdot\sqrt[4]{34+\frac{13}{18}}=\sqrt[4]{2812+\frac{1}{2}}}}](/mediawiki/images/math/f/7/0/f704a237c9fcf421bb4cd38ac8726658.png)
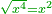

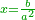
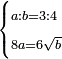

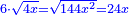

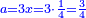
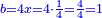
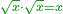

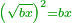

![\scriptstyle{\color{OliveGreen}{x=\sqrt[3]{\frac{b}{a^2}}}}](/mediawiki/images/math/e/8/1/e8184d1bbdd07b6234395c96f79f46ec.png)
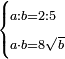

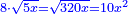
![\scriptstyle{\color{blue}{x=\sqrt[3]{\frac{320}{10^2}}=\sqrt[3]{\frac{320}{100}}=\sqrt[3]{3+\frac{1}{5}}}}](/mediawiki/images/math/a/8/5/a85d6aba64f34f32b4d18eaec2fc80c1.png)
![\scriptstyle{\color{blue}{a=2x=2\sdot\sqrt[3]{3+\frac{1}{5}}=\sqrt[3]{25+\frac{3}{5}}}}](/mediawiki/images/math/d/1/0/d10e124a3ed9a20e599d0fbe1563ddcf.png)
![\scriptstyle{\color{blue}{b=5x=5\sdot\sqrt[3]{3+\frac{1}{5}}=\sqrt[3]{400}}}](/mediawiki/images/math/a/c/e/aceba9004b938a10d9b256e95a97df27.png)
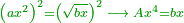
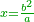
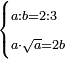
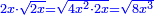

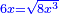
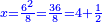

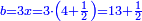
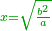
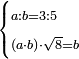
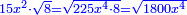
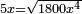

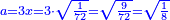
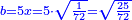

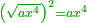
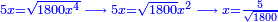
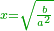
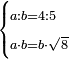
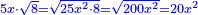
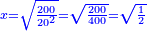

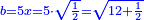

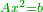 .
.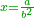
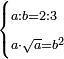
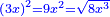

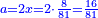
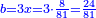
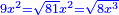



![\scriptstyle{\color{OliveGreen}{x=\sqrt[4]{\frac{b}{a^2}}}}](/mediawiki/images/math/4/a/c/4acdc67ddf5b5244591dbe4ffb0a9ea0.png)
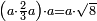
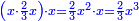
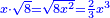
![\scriptstyle{\color{blue}{x=\sqrt[4]{\frac{8}{\frac{2}{3}^2}}=\sqrt[4]{\frac{8}{\frac{4}{9}}}=\sqrt[4]{18}}}](/mediawiki/images/math/1/e/2/1e20eb7ec843d568d70183b754cc3d9a.png)
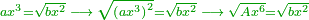
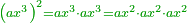
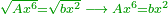
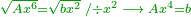
![\scriptstyle{\color{OliveGreen}{x=\sqrt[6]{\frac{b}{a^2}}}}](/mediawiki/images/math/3/5/c/35ceb2c7284ec62f2528e0a7aa41bed4.png)
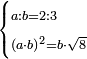
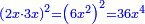
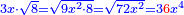
![\scriptstyle{\color{blue}{x=\sqrt[6]{\frac{72}{36^2}}=\sqrt[6]{\frac{72}{1296}}=\sqrt[6]{\frac{1}{18}}}}](/mediawiki/images/math/9/7/a/97a8cee017540ff4db61c686548d1193.png)
![\scriptstyle{\color{blue}{a=2x=2\sdot\sqrt[6]{\frac{1}{18}}=\sqrt[6]{\frac{64}{18}}=\sqrt[6]{3+\frac{5}{9}}}}](/mediawiki/images/math/6/b/6/6b6891ece57619216a2e240f9749d4a3.png)
![\scriptstyle{\color{blue}{b=3x=3\sdot\sqrt[6]{\frac{1}{18}}=\sqrt[6]{\frac{729}{18}}=\sqrt[6]{40+\frac{1}{2}}}}](/mediawiki/images/math/5/3/1/531725e648b9b626aca7b317fb3f9b78.png)
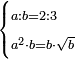

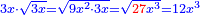
![\scriptstyle{\color{blue}{x=\sqrt[3]{\frac{{\color{red}{27}}}{12^2}}=\sqrt[3]{\frac{{\color{red}{27}}}{144}}=\sqrt[3]{\frac{3}{16}}}}](/mediawiki/images/math/f/b/8/fb8b435e92545e6e22a069fdad8bc6e3.png)
![\scriptstyle{\color{blue}{a=2x=2\sdot\sqrt[3]{\frac{3}{16}}=\sqrt[3]{1+\frac{1}{2}}}}](/mediawiki/images/math/a/b/2/ab2036f2bf8ea4c40af41393d46c67a0.png)
![\scriptstyle{\color{blue}{b=3x=3\sdot\sqrt[3]{\frac{3}{16}}=\sqrt[3]{{\color{red}{5+\frac{1}{16}}}}}}](/mediawiki/images/math/3/1/a/31a491bf029100829c36ce4e7d6aa252.png)
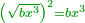
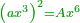
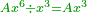

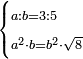
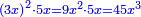
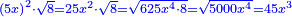

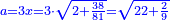
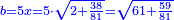
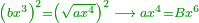
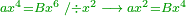



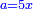 ] and the other is seven things [
] and the other is seven things [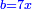 ]
]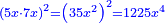

![\scriptstyle{\color{blue}{x=\sqrt[4]{\frac{5000}{1225^2}}=\sqrt[4]{\frac{5000}{1500625}}=\sqrt[4]{\frac{8}{2401}}}}](/mediawiki/images/math/2/c/6/2c6d6086b1218d50d8efa89b91969bf2.png)
![\scriptstyle{\color{blue}{a=5x=5\sdot\sqrt[4]{\frac{8}{2401}}=\sqrt[4]{2+\frac{198}{2401}}}}](/mediawiki/images/math/9/5/4/9546761c92c86062bfc246cdc8b1b834.png)
![\scriptstyle{\color{blue}{b=7x=7\sdot\sqrt[4]{\frac{8}{2401}}=\sqrt[4]{8}}}](/mediawiki/images/math/7/a/6/7a68ae09bae5347e5cf55f85337c9f8c.png)

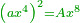
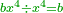
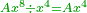
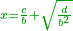
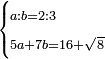
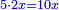
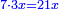
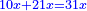

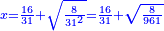

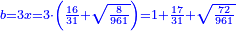

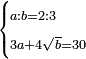


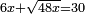
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle x&\scriptstyle=\left(\sqrt{\frac{30}{6}+\left(\frac{1}{4}\sdot\frac{48}{6^2}\right)}-\sqrt{\frac{1}{4}\sdot\frac{48}{6^2}}\right)^2=\left(\sqrt{5+\left(\frac{1}{4}\sdot\frac{48}{36}\right)}-\sqrt{\frac{1}{4}\sdot\frac{48}{36}}\right)^2=\left[\sqrt{5+\left[\frac{1}{4}\sdot\left(1+\frac{1}{3}\right)\right]}-\sqrt{\frac{1}{4}\sdot\left(1+\frac{1}{3}\right)}\right]^2\\&\scriptstyle=\left(\sqrt{5+\frac{1}{3}}-\sqrt{\frac{1}{3}}\right)^2=5+\frac{2}{3}-\sqrt{\frac{64}{9}}=5+\frac{2}{3}-\left(2+\frac{2}{3}\right)=3\\\end{align}}}](/mediawiki/images/math/9/2/e/92e11074315519b721cc663224d5dc3e.png)
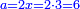

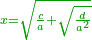

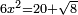
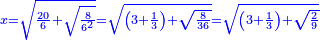
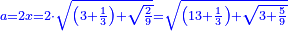
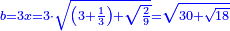
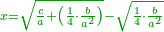
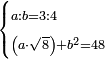
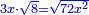
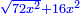
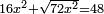
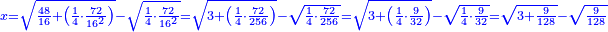
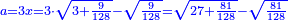

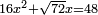
![\scriptstyle{\color{OliveGreen}{x=\sqrt[3]{\frac{c}{a}+\sqrt{\frac{d}{a^2}}}}}](/mediawiki/images/math/3/6/0/3602d816e0d45d8dc5458c5389874675.png)
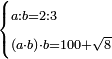


![\scriptstyle{\color{blue}{\begin{align}\scriptstyle x&\scriptstyle=\sqrt[3]{\frac{100}{18}+\sqrt{\frac{8}{18^2}}}\\&\scriptstyle=\sqrt[3]{\left(5+\frac{5}{9}\right)+\sqrt{\frac{8}{324}}}\\&\scriptstyle=\sqrt[3]{\left(5+\frac{5}{9}\right)+\sqrt{\frac{2}{81}}}\\\end{align}}}](/mediawiki/images/math/c/2/f/c2f3b4ce2eec2b96e16e0ae50a912270.png)
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle a=2x&\scriptstyle=2\sdot\sqrt[3]{\left(5+\frac{5}{9}\right)+\sqrt{\frac{2}{81}}}\\&\scriptstyle=\sqrt[3]{8\sdot\left[\left(5+\frac{5}{9}\right)+\sqrt{\frac{2}{81}}\right]}\\&\scriptstyle=\sqrt[3]{\left(44+\frac{4}{9}\right)+\sqrt{1+\frac{47}{81}}}\\\end{align}}}](/mediawiki/images/math/c/a/0/ca0ac6d7e56d5e598aed5863fe2f1072.png)
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle b=3x&\scriptstyle=3\sdot\sqrt[3]{\left(5+\frac{5}{9}\right)+\sqrt{\frac{2}{81}}}\\&\scriptstyle=\sqrt[3]{\left[27\sdot\left(5+\frac{5}{9}\right)\right]+\sqrt{27^2\sdot\frac{2}{81}}}\\&\scriptstyle=\sqrt[3]{150+\sqrt{729\sdot\frac{2}{81}}}\\&\scriptstyle=\sqrt[3]{150+\sqrt{18}}\\\end{align}}}](/mediawiki/images/math/2/6/7/26703f09836b0e76113e56eb12ac7c5b.png)
![\scriptstyle{\color{OliveGreen}{x=\sqrt[3]{\left(\sqrt{\frac{c}{a}+\left(\frac{1}{4}\sdot\frac{b}{a^2}\right)}-\sqrt{\frac{1}{4}\sdot\frac{b}{a^2}}\right)^2}}}](/mediawiki/images/math/7/1/8/7189d55dca8313496388a4cf2ad7ec31.png)

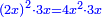
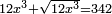
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle x&\scriptstyle=\sqrt[3]{\left(\sqrt{\frac{342}{12}+\left(\frac{1}{4}\sdot\frac{12}{12^2}\right)}-\sqrt{\frac{1}{4}\sdot\frac{12}{12^2}}\right)^2}\\&\scriptstyle=\sqrt[3]{\left(\sqrt{\left(28+\frac{1}{2}\right)+\left(\frac{1}{4}\sdot\frac{12}{144}\right)}-\sqrt{\frac{1}{4}\sdot\frac{12}{144}}\right)^2}\\&\scriptstyle=\sqrt[3]{\left(\sqrt{\left(28+\frac{1}{2}\right)+\left(\frac{1}{4}\sdot\frac{1}{12}\right)}-\sqrt{\frac{1}{4}\sdot\frac{1}{12}}\right)^2}\\&\scriptstyle=\sqrt[3]{\left(\sqrt{\left(28+\frac{1}{2}\right)+\frac{1}{48}}-\sqrt{\frac{1}{48}}\right)^2}\\&\scriptstyle=\sqrt[3]{\left(\sqrt{28+\frac{25}{48}}-\sqrt{\frac{1}{48}}\right)^2}=\sqrt[3]{27}=3\\\end{align}}}](/mediawiki/images/math/2/2/d/22d4582387a4a3c140a3e933473288cb.png)
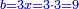
![\scriptstyle{\color{OliveGreen}{x=\sqrt[4]{\frac{c}{a}+\sqrt{\frac{d}{a^2}}}}}](/mediawiki/images/math/e/b/1/eb1d4e746d7792772dc58303c43553d2.png)
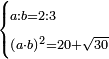
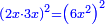
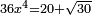
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle x&\scriptstyle=\sqrt[4]{\frac{20}{36}+\sqrt{\frac{30}{36^2}}}\\&\scriptstyle=\sqrt[4]{\frac{5}{9}+\sqrt{\frac{30}{1296}}}\\&\scriptstyle=\sqrt[4]{\frac{5}{9}+\sqrt{\frac{5}{216}}}\\\end{align}}}](/mediawiki/images/math/2/2/c/22ca5b04f6690920f294e4b60b95ad06.png)
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle a=2x&\scriptstyle=2\sdot\sqrt[4]{\frac{5}{9}+\sqrt{\frac{5}{216}}}\\&\scriptstyle=\sqrt[4]{\left(8+\frac{8}{9}\right)+\sqrt{5+\frac{25}{27}}}\\\end{align}}}](/mediawiki/images/math/2/f/e/2fe633ca04c68c7e292f70eb11bf8401.png)
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle b=3x&\scriptstyle=3\sdot\sqrt[4]{\frac{5}{9}+\sqrt{\frac{5}{216}}}\\&\scriptstyle=\sqrt[4]{45+\sqrt{151+\frac{189}{216}}}\\\end{align}}}](/mediawiki/images/math/c/d/2/cd2d416a195f8734b85ba0de1137a839.png)
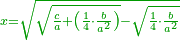
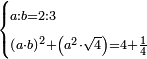
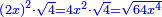

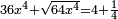
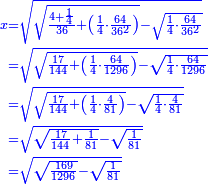
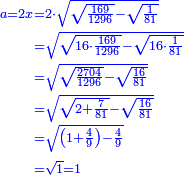

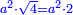
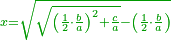
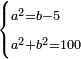
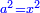

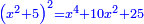
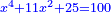
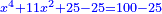
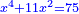

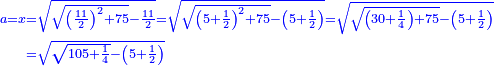
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle b=x^2+5&\scriptstyle=\left[\sqrt{\sqrt{105+\frac{1}{4}}-\left(5+\frac{1}{2}\right)}\right]^2+5=\sqrt{105+\frac{1}{4}}-\left(5+\frac{1}{2}\right)+5\\&\scriptstyle=\sqrt{105+\frac{1}{4}}-\frac{1}{2}\\\end{align}}}](/mediawiki/images/math/a/a/8/aa868a065f0e07f23d85ac3d2965d30f.png)
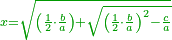
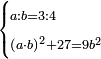
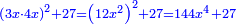
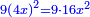
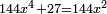
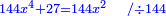
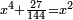
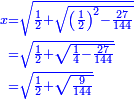
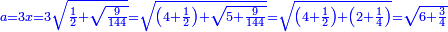
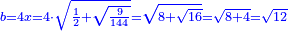
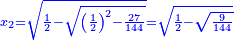
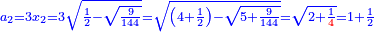
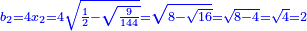

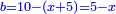 ].
].![\scriptstyle{\color{blue}{\left[\left(x+5\right)-\left(5-x\right)\right]^2\sdot\left(7+\frac{1}{9}\right)=\left(2x\right)^2\sdot\left(7+\frac{1}{9}\right)=4x^2\sdot\left(7+\frac{1}{9}\right)=\left(28+\frac{4}{9}\right)x^2}}](/mediawiki/images/math/1/5/1/151b42d3ed2a00135bd225e80ab702b6.png)
![\scriptstyle{\color{blue}{\left[\left(x+5\right)\sdot\left(5-x\right)\right]^2=\left(25-x^2\right)^2}}](/mediawiki/images/math/a/9/c/a9c85f85202d807343a660770338f30e.png)
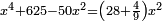

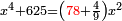

![\scriptstyle{\color{blue}{\begin{align}\scriptstyle x&\scriptstyle=\sqrt{\left[\frac{1}{2}\sdot\left(78+\frac{4}{9}\right)\right]-\sqrt{\left[\frac{1}{2}\sdot\left(78+\frac{4}{9}\right)\right]^2-625}}=\sqrt{\left(39+\frac{2}{9}\right)-\sqrt{\left(39+\frac{2}{9}\right)^2-625}}\\&\scriptstyle=\sqrt{\left(39+\frac{2}{9}\right)-\sqrt{\left(1538+\frac{31}{81}\right)-625}}=\sqrt{\left(39+\frac{2}{9}\right)-\sqrt{913+\frac{31}{81}}}\\\end{align}}}](/mediawiki/images/math/e/3/8/e38041d48a7ba6f299624edd33a3eb76.png)

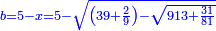
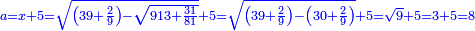

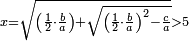

![\scriptstyle\begin{cases}\scriptstyle a+b=10\\\scriptstyle\left[\left(a\sdot b\right)+a^2\right]\sdot a=\left[a\sdot\left(\frac{1}{2}a\right)\right]^2+36\end{cases}](/mediawiki/images/math/5/2/b/52b64b95ed608c25a1c3a780e3cd0c7a.png)
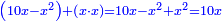

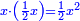

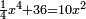
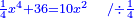
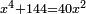
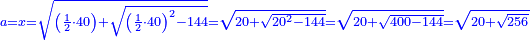
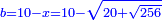

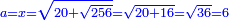
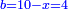
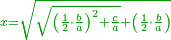
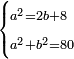
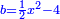 ].
].
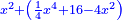
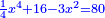
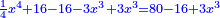
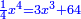
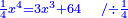



![\scriptstyle bx=\sqrt[3]{c}](/mediawiki/images/math/4/e/c/4eced3ba1c8f51bf47cf911659b6e201.png)
![\scriptstyle{\color{OliveGreen}{x=\sqrt[3]{\frac{c}{b^3}}}}](/mediawiki/images/math/b/5/8/b58f8866507ea6bbb9f8ab2a606fa01a.png)
![\scriptstyle\begin{cases}\scriptstyle a:b=2:3\\\scriptstyle3a+4b=\sqrt[3]{216}\end{cases}](/mediawiki/images/math/3/7/c/37c574e931d2b293ce8e7c05872dd409.png)

![\scriptstyle{\color{blue}{6x+12x=18x=\sqrt[3]{216}}}](/mediawiki/images/math/c/e/b/ceb776e4b0edd69a32ac80b8bc337f44.png)
![\scriptstyle{\color{blue}{x=\sqrt[3]{\frac{216}{18^3}}=\sqrt[3]{\frac{216}{18^2\sdot18}}=\sqrt[3]{\frac{216}{324\sdot18}}=\sqrt[3]{\frac{216}{5832}}=\sqrt[3]{\frac{1}{27}}}}](/mediawiki/images/math/9/4/8/9484cfee86010edc99a4a5c975ad6f3b.png)
![\scriptstyle{\color{blue}{a=2x=2\sdot\sqrt[3]{\frac{1}{27}}=\sqrt[3]{\frac{8}{27}}=\frac{2}{3}}}](/mediawiki/images/math/0/3/8/0382c9bb4fcf3ce484bd047833a28740.png)
![\scriptstyle{\color{blue}{b=3x=3\sdot\sqrt[3]{\frac{1}{27}}=\sqrt[3]{\frac{27}{27}}=\sqrt[3]{1}=1}}](/mediawiki/images/math/7/d/8/7d8f893f3a3080698fb59da107eeea37.png)

![\scriptstyle\begin{cases}\scriptstyle a:b=3:4\\\scriptstyle\sqrt[3]{2a+3b}=8\end{cases}](/mediawiki/images/math/f/d/f/fdfeea3918f71a57781b72d247caef5c.png)

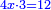
![\scriptstyle{\color{blue}{\sqrt[3]{18x}=8}}](/mediawiki/images/math/3/d/5/3d50a9d323ad1b6f10c2db111215a6d0.png)
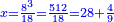
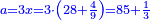
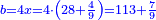
![\scriptstyle{\color{OliveGreen}{\sqrt[3]{x}\sdot\sqrt[3]{x}=\sqrt[3]{x^2}}}](/mediawiki/images/math/e/1/7/e17348676259e603862284a259e81ae0.png)
![\scriptstyle{\color{OliveGreen}{\sqrt[3]{x^2}\sdot\sqrt[3]{x}=\sqrt[3]{x^3}=x}}](/mediawiki/images/math/3/3/7/3374ceeebcdbd1fed96cb0a5116663f1.png)
![\scriptstyle{\color{OliveGreen}{x=\sqrt[6]{\frac{c}{a^3}}}}](/mediawiki/images/math/d/1/b/d1b43e5c0bc13bfbef4f7f999b76fbe9.png)
![\scriptstyle\begin{cases}\scriptstyle a:b=3:5\\\scriptstyle a\sdot b=\sqrt[3]{729}\end{cases}](/mediawiki/images/math/0/5/a/05a756d12f1bd790b4653042daf26458.png)
![\scriptstyle{\color{blue}{3x\sdot5x=15x^2=\sqrt[3]{729}}}](/mediawiki/images/math/b/1/7/b17a20334928eeb85eb7b11e63e6e5b5.png)
![\scriptstyle{\color{blue}{x=\sqrt[6]{\frac{729}{15^3}}=\sqrt[6]{\frac{729}{3375}}=\sqrt[6]{\frac{27}{125}}}}](/mediawiki/images/math/8/2/1/821350bd6eed5dd5a71a0cd2181ecce0.png)
![\scriptstyle{\color{blue}{a=3x=3\sdot\sqrt[6]{\frac{27}{125}}=\sqrt[6]{1{\color{red}{57}}+\frac{58}{125}}=\sqrt{5+\frac{2}{5}}}}](/mediawiki/images/math/6/e/0/6e0d6bc95cc0a0b61205e6c95fd75260.png)
![\scriptstyle{\color{blue}{b=5x=5\sdot\sqrt[6]{\frac{27}{125}}=\sqrt[6]{3375}=\sqrt{15}}}](/mediawiki/images/math/5/1/f/51f328d09bbb5fa38b5a7a64972bdf6a.png)
![\scriptstyle{\color{OliveGreen}{ax^2=\sqrt[3]{c}\longrightarrow ax^3=\sqrt{c}}}](/mediawiki/images/math/1/d/c/1dc626e57646e31cfe6c1a66516c2af7.png)
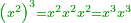
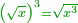
![\scriptstyle{\color{OliveGreen}{x^2=\sqrt[3]{c}\longrightarrow\left(x^2\right)^3=c}}](/mediawiki/images/math/0/e/2/0e20995fabafb503ba1cf5ef72bd3237.png)
![\scriptstyle{\color{OliveGreen}{x^2=\sqrt[3]{c}\;\longleftrightarrow\;x^6=c\;\longleftrightarrow\;x^3=\sqrt{c}}}](/mediawiki/images/math/1/c/1/1c144bd872b01ecd908732d86ccc1d50.png)
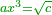

![\scriptstyle\begin{cases}\scriptstyle a:b=1:3\\\scriptstyle\sqrt[3]{a}\sdot\sqrt[3]{b}=100\end{cases}](/mediawiki/images/math/6/b/6/6b684d922156985988350c4b2a4c248d.png)
![\scriptstyle{\color{blue}{\sqrt[3]{x}\sdot\sqrt[3]{3x}=\sqrt[3]{3x^2}=100}}](/mediawiki/images/math/7/2/d/72d1c1182dc0dac8f572d1b8f402b828.png)

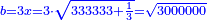
![\scriptstyle{\color{OliveGreen}{\sqrt[3]{c}=\sqrt[3]{ax^2}\;\longleftrightarrow\;c^3=ax^2}}](/mediawiki/images/math/f/f/9/ff95f8cbf7beec0010660015633f4d1b.png)
![\scriptstyle{\color{OliveGreen}{x=\sqrt[9]{\frac{c}{a^3}}}}](/mediawiki/images/math/2/1/b/21bcf6d5386debf7b777f5961c25ae36.png)
![\scriptstyle\begin{cases}\scriptstyle a:b=1:4\\\scriptstyle\left(a\sdot b\right)\sdot a=\sqrt[3]{216}\end{cases}](/mediawiki/images/math/1/7/1/171ce646462d735ef90bd02638d35efb.png)

![\scriptstyle{\color{blue}{4x^2\sdot x=4x^3=\sqrt[3]{216}}}](/mediawiki/images/math/e/b/f/ebfe0ac5dcb37217e05ca762bf4c010f.png)
![\scriptstyle{\color{blue}{a=x=\sqrt[9]{\frac{216}{4^3}}=\sqrt[9]{\frac{216}{64}}=\sqrt[9]{3+\frac{3}{8}}}}](/mediawiki/images/math/d/f/e/dfed5d3354610a31339918a8491667b6.png)
![\scriptstyle{\color{blue}{b=4x=4\sdot\sqrt[9]{3+\frac{3}{8}}=\sqrt[9]{262144\sdot\left(3+\frac{3}{8}\right)}=\sqrt[9]{884736}}}](/mediawiki/images/math/3/7/f/37f6815dd0501455ae2f0e6c0e2131b9.png)