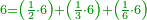Difference between revisions of "ספר חשבון"
(→Find a Quantity Problem - First from Last) |
(→Divisibility of the Numbers 1-112 (MS Ithaca)) |
||
| (395 intermediate revisions by 2 users not shown) | |||
| Line 1: | Line 1: | ||
| − | + | {{#annotpage: time="1400-1500", peshat_title="00002042"}} | |
__TOC__ | __TOC__ | ||
| − | + | ||
{| | {| | ||
| + | |- | ||
| + | |Arithmetic Book | ||
| + | |style="width:45%;text-align:right;"|‫<ref>31v</ref><big>ספר חשבון</big> | ||
|- | |- | ||
| | | | ||
| − | == The Nature of One == | + | == <span style=color:green>The Nature of One</span> == |
| | | | ||
|- | |- | ||
| − | |One | + | |One, from one aspect, is a number and the foundation of the numbers; from another aspect it is not a number. |
| − | |style="text-align:right;"|האחד מדרך אחד<ref>אחד: MS Cambridge א‫'< | + | |style="text-align:right;"|<big>האחד מדרך אחד הוא מן המספר</big> ועיקר המספר ומדרך אחר אינו מספר‫<ref>MS Cambridge: title is missing<br> |
| + | אחד: MS Cambridge א‫' MS Ithaca חשבון<br> | ||
| + | מן המספר: MS Cambridge מספר; Ithaca: המספר ומדרך א<br> | ||
| + | ועיקר המספר: MS Cambridge om.</ref> | ||
| + | |- | ||
| + | |The proof that indicates that it is not a number is that every number is half [the sum of the numbers on] its both sides and also [the sum of the numbers on] the sides of its both sides and so on until the end of its sides. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{n=\frac{1}{2}\sdot\left[\left(n-a\right)+\left(n+a\right)\right]}}</math> | ||
| + | |style="text-align:right;"|והאות המורה כי אננו מספר הוא כי כל מספר הוא מחצית שני צדיו וכן הוא מחצית שני צדי צדיו על תכלית כל צדיו‫<ref>הוא: MS Cambridge om.<br> | ||
| + | על: MS Cambridge עד</ref> | ||
| + | |- | ||
| + | | | ||
| + | :Example: 10 - its one side is 11 and its other side is 9; together they are 20 and 10 is half [the sum of] both sides. | ||
| + | :<math>\scriptstyle{\color{blue}{10=\frac{1}{2}\sdot20=\frac{1}{2}\sdot\left(9+11\right)}}</math> | ||
| + | |style="text-align:right;"|המשל בזה י' צדו האחד י"א וצדו האחר ט' ושניהם כ' וי' הוא חצי שני הצדדים‫<ref>הצדדים: MS Ithaca צדי הצדדים</ref> | ||
|- | |- | ||
| | | | ||
| − | + | :It is also half [the sum of the numbers on] the sides of its sides, which are 12 and 8. | |
| − | :<math>\scriptstyle | + | :<math>\scriptstyle{\color{blue}{10=\frac{1}{2}\sdot\left(8+12\right)}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכן הוא חצי שני צדי צדיו שהם י"ב וח‫' |
|- | |- | ||
| | | | ||
| − | : | + | :It is also half [the sum of the numbers on] the sides of the sides of its sides, which are 13 and 7. |
| − | + | :<math>\scriptstyle{\color{blue}{10=\frac{1}{2}\sdot\left(7+13\right)}}</math> | |
| − | + | |style="text-align:right;"|וכן הוא מחצית שני צדי צדי צדיו שהם י"ג וז‫'‫<ref>צדי: MS Ithaca om.</ref> | |
| − | |style="text-align:right;"| | + | |- |
| − | + | |Ans so on, every number is half [the sum of the numbers on] its both sides. | |
| − | וכן הוא מחצית שני צדי צדי צדיו שהם י"ג וז‫'< | + | |style="text-align:right;"|וכן כל המספר הוא מחצית ב' צידיו |
| − | וכן כל | ||
|- | |- | ||
| | | | ||
| − | : | + | :Since one is not a number, you do not find two sides of it. |
| − | |style="text-align:right;"|והאחד מפני שאינו<ref>שאינו: MS Cambridge שהוא אינו</ref> | + | |style="text-align:right;"|והאחד מפני שאינו מספר אי אתה מוצא לו ב' צדדים‫<ref>שאינו: MS Cambridge שהוא אינו</ref> |
|- | |- | ||
| | | | ||
| − | : | + | :Since it is a number, it is half [the number on] its one side, i.e. half the number 2. |
| − | |style="text-align:right;"|ומצד היותו מספר הוא חצי צדו האחד ר"ל חצי מספר ב‫' | + | :<math>\scriptstyle{\color{blue}{1=\frac{1}{2}\sdot2}}</math> |
| + | |style="text-align:right;"|ומצד היותו מספר הוא מחצית חצי צדו האחד ר"ל חצי מספר ב‫' | ||
| + | |- | ||
| + | |Another proof that indicates that one is not a number is that every number is either integer or a fraction. | ||
| + | |style="text-align:right;"|<big>ואות אחר</big> מורה שהאחד אינו מספר כי כל מספ' הוא או מספר שלם או שבר מספר‫<ref>מורה: MS Cambridge מוראה<br> | ||
| + | שבר: MS Ithaca <s>חצי</s> marg. שבר</ref> | ||
|- | |- | ||
| | | | ||
| − | * | + | *The property of the integer is that when you multiply it by itself it increases. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{OliveGreen}{n>1\longrightarrow n<n\times n}}</math> |
| + | |style="text-align:right;"|ומדרך המספר השלם כשתרבה אותו בעצמו יוסיף | ||
|- | |- | ||
| | | | ||
| − | : | + | ::As 2 times 2, which is 4. |
| − | ::<math>\scriptstyle | + | ::<math>\scriptstyle{\color{blue}{2<2\times2=4}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כגון ב' פעמי' ב' ד‫'‫<ref>ב'פ'ב‫'‫ MS Cambridge :ב' פעמי' ב‫'</ref> |
|- | |- | ||
| | | | ||
| − | :::<math>\scriptstyle{\color{blue}{ | + | ::3 times 3, which is 9. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{3<3\times3=9}}</math> |
| + | |style="text-align:right;"|ג' פעמי' ג' ט‫'‫<ref>ג'פ'ג‫'‫ MS Cambridge :ג' פעמי' ג‫'</ref> | ||
|- | |- | ||
| | | | ||
| − | : | + | :And so on. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכן כלם |
|- | |- | ||
| | | | ||
| − | + | *Whereas [the property of] the fraction is that when you multiply it by itself it decreases. | |
| − | + | :<math>\scriptstyle{\color{OliveGreen}{0<n<1\longrightarrow n\times n<n}}</math> | |
|style="text-align:right;"|ושבר מספר כשתרבה<ref>כשתרבה: MS Cambridge כשאתה מרבה</ref> אותו בעצמו יחסר | |style="text-align:right;"|ושבר מספר כשתרבה<ref>כשתרבה: MS Cambridge כשאתה מרבה</ref> אותו בעצמו יחסר | ||
|- | |- | ||
| | | | ||
| − | :::<math>\scriptstyle{\color{blue}{\frac{1}{4}=\frac{1}{2}\times\frac{1}{2}<\frac{1}{2}}}</math> | + | ::As half times a half, which is a quarter. |
| + | ::<math>\scriptstyle{\color{blue}{\frac{1}{4}=\frac{1}{2}\times\frac{1}{2}<\frac{1}{2}}}</math> | ||
|style="text-align:right;"|כגון<ref>כגון: MS Cambridge כמו</ref> חצי פעם חצי<ref>חצי פעם חצי: MS Cambridge חצי פעם חצי פעם</ref> שהוא רביע | |style="text-align:right;"|כגון<ref>כגון: MS Cambridge כמו</ref> חצי פעם חצי<ref>חצי פעם חצי: MS Cambridge חצי פעם חצי פעם</ref> שהוא רביע | ||
|- | |- | ||
| | | | ||
| − | :::<math>\scriptstyle{\color{blue}{\frac{1}{9}=\frac{1}{3}\times\frac{1}{3}<\frac{1}{3}}}</math> | + | ::A third times a third, which is a ninth. |
| − | |style="text-align:right;"|שליש פעם שליש<ref>שליש פעם שליש: MS Cambridge שליש פעם שליש פעם</ref> שהוא<ref>שהוא: MS Cambridge הוא</ref> תשיע | + | ::<math>\scriptstyle{\color{blue}{\frac{1}{9}=\frac{1}{3}\times\frac{1}{3}<\frac{1}{3}}}</math> |
| + | |style="text-align:right;"|שליש פעם א' שליש<ref>שליש פעם שליש: MS Cambridge שליש פעם שליש פעם</ref> שהוא<ref>שהוא: MS Cambridge הוא</ref> תשיע | ||
|- | |- | ||
| | | | ||
| − | :::<math>\scriptstyle{\color{blue}{\frac{1}{16}=\frac{1}{4}\times\frac{1}{4}<\frac{1}{4}}}</math> | + | ::A quarter times a quarter, which is a part of 16. |
| − | |style="text-align:right;"|רביע פעם רביע<ref>רביע פעם רביע: MS Cambridge רביע פעם רביע פעם</ref> שהוא<ref>שהוא: MS Cambridge הוא</ref> חלק מי"ו | + | ::<math>\scriptstyle{\color{blue}{\frac{1}{16}=\frac{1}{4}\times\frac{1}{4}<\frac{1}{4}}}</math> |
| + | |style="text-align:right;"|רביע פעם רביע<ref>רביע פעם רביע: MS Cambridge רביע פעם רביע פעם</ref> שהוא<ref>שהוא: MS Cambridge הוא</ref> חלק מי"ו | ||
|- | |- | ||
| | | | ||
| − | : | + | :And so on. |
| − | |style="text-align:right;"|והאחד שאינו מוסיף על חשבונו כשתמנהו בעצמו כדרך השלמים ולא גורע ממניינו כדרך השברים יורה שאיננו<ref>שאיננו: MS Cambridge כי אינו</ref> מספר | + | |style="text-align:right;"|וכן כלם |
| − | + | |- | |
| − | + | | | |
| + | :Since one does not increase when you multiply it by itself, as the integers, and does not decrease, as the fractions, this indicates that it is not a number. | ||
| + | :<math>\scriptstyle{\color{blue}{1=1\times1}}</math> | ||
| + | |style="text-align:right;"|<big>והאחד</big> שאינו מוסיף על חשבונו כשתמנהו בעצמו כדרך השלמים ולא גורע [ממניינו]‫<ref>MS Ithaca om.</ref> כדרך השברים יורה שאיננו‫<ref>שאיננו: MS Cambridge כי אינו</ref> מספר | ||
|- | |- | ||
| | | | ||
| − | == Shortcuts - Products of Ranks by Ranks == | + | == <span style=color:green>Shortcuts - Products of Ranks by Ranks</span> == |
| | | | ||
|- | |- | ||
| + | !<span style=color:green>Units by Tens</span> | ||
| | | | ||
| − | + | |- | |
| − | :<math>\scriptstyle a\times\left(10\sdot b\right)</math> | + | |When we want to multiply units by tens. |
| − | |style="text-align:right;"|כשנרצה<ref>כשנרצה: MS Cambridge ואם נרצה</ref> לרבות<ref>לרבות: MS Cambridge לדעת</ref> אחדים עם עשרות | + | :<math>\scriptstyle{\color{OliveGreen}{a\times\left(10\sdot b\right)}}</math> |
| + | |style="text-align:right;"|<big>כשנרצה<ref>כשנרצה: MS Cambridge ואם נרצה</ref> לרבות<ref>לרבות: MS Cambridge לדעת</ref> אחדים</big> עם עשרות | ||
|- | |- | ||
| | | | ||
| − | : | + | *As 6 times 40. |
| + | :<math>\scriptstyle6\times40</math> | ||
|style="text-align:right;"|כגון ו' פעמי' מ‫' | |style="text-align:right;"|כגון ו' פעמי' מ‫' | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{6\times40=\left(6\sdot4\right)\sdot10=24\sdot10=240}}</math> | + | :We take their analogous from the corresponding units: since 40 is 4 tens multiplied 6 times, and since 4 times 6 is 24, then 6 times 40 is 24 tens, which is 240. |
| + | :<math>\scriptstyle{\color{blue}{6\times40=\left(6\sdot4\right)\sdot10=24\sdot10=240}}</math> | ||
|style="text-align:right;"|נקח<ref>נקח: MS Cambridge om.</ref> דמיונם<ref>דמיונם: MS Cambridge שדמיונם</ref> מן האחדים הדומים להם ובעבור שמ' הם ד' עשרות מניינם הוא ו' פעמים וכמו<ref>מן ... וכמו: MS Cambridge om.</ref> שו' פעמי' ד'<ref>ו'פ'ד‫'‫ MS Cambridge :שו' פעמי' ד‫'</ref> הם<ref>הם: MS Cambridge שהם</ref> כ"ד כן ו' פעמים מ'<ref>‫כן ... מ‫': MS Cambridge om.</ref> הם<ref>הם: MS Cambridge בין יהיו</ref> כ"ד עשרות שהם ר"מ | |style="text-align:right;"|נקח<ref>נקח: MS Cambridge om.</ref> דמיונם<ref>דמיונם: MS Cambridge שדמיונם</ref> מן האחדים הדומים להם ובעבור שמ' הם ד' עשרות מניינם הוא ו' פעמים וכמו<ref>מן ... וכמו: MS Cambridge om.</ref> שו' פעמי' ד'<ref>ו'פ'ד‫'‫ MS Cambridge :שו' פעמי' ד‫'</ref> הם<ref>הם: MS Cambridge שהם</ref> כ"ד כן ו' פעמים מ'<ref>‫כן ... מ‫': MS Cambridge om.</ref> הם<ref>הם: MS Cambridge בין יהיו</ref> כ"ד עשרות שהם ר"מ | ||
|- | |- | ||
| | | | ||
| − | : | + | *The same for 3 times 30. |
| + | :<math>\scriptstyle3\times30</math> | ||
|style="text-align:right;"|וכן<ref>וכן: MS Cambridge <s>וג'</s> וכן</ref> ג' פעמים ל‫'<ref>ג'פ'ל‫'‫ MS Cambridge :ג' פעמים ל‫'</ref> | |style="text-align:right;"|וכן<ref>וכן: MS Cambridge <s>וג'</s> וכן</ref> ג' פעמים ל‫'<ref>ג'פ'ל‫'‫ MS Cambridge :ג' פעמים ל‫'</ref> | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{3\times30=\left(3\sdot3\right)\sdot10=9\sdot10=90}}</math> | + | :Their analogous is 3 times 3, which is 9. So, it is 9 tens, which is 90. |
| − | |style="text-align:right;"|דמיונם הוא<ref>הוא: MS Cambridge om.</ref> ג' פעמים ג' שהם ט' ויהיו<ref>ויהיו: MS Cambridge כן ג'פ'ל' יהיו</ref> ט' עשרות שהם צ' ועל זה הדרך הם כלם‫<ref>כשנרצה ... כלם: MS Cambridge the discussion on multiplication of units by tens appears after the discussion on multiplication of tens by tens</ref> | + | :<math>\scriptstyle{\color{blue}{3\times30=\left(3\sdot3\right)\sdot10=9\sdot10=90}}</math> |
| + | |style="text-align:right;"|דמיונם הוא<ref>הוא: MS Cambridge om.</ref> ג' פעמים ג' שהם ט' ויהיו<ref>ויהיו: MS Cambridge כן ג'פ'ל' יהיו</ref> ט' עשרות שהם צ‫' | ||
| + | |- | ||
| + | |This way for all of them. | ||
| + | |style="text-align:right;"|ועל זה הדרך הם כלם‫<ref>כשנרצה ... כלם: MS Cambridge the discussion on multiplication of units by tens appears after the discussion on multiplication of tens by tens</ref> | ||
|- | |- | ||
| + | !<span style=color:green>Tens by Tens</span> | ||
| | | | ||
| − | + | |- | |
| − | :<math>\scriptstyle\left(a\sdot10\right)\times\left(10\sdot b\right)</math> | + | |If we multiply tens by tens. |
| + | :<math>\scriptstyle{\color{OliveGreen}{\left(a\sdot10\right)\times\left(10\sdot b\right)}}</math> | ||
|style="text-align:right;"|ואם נרבה<ref>ואם נרבה: MS Cambridge כשתרבה</ref> עשרות עם עשרות | |style="text-align:right;"|ואם נרבה<ref>ואם נרבה: MS Cambridge כשתרבה</ref> עשרות עם עשרות | ||
|- | |- | ||
| | | | ||
| − | : | + | *As 30 times 30. |
| + | :<math>\scriptstyle30\times30</math> | ||
|style="text-align:right;"|כגון ל' פעמים ל‫' | |style="text-align:right;"|כגון ל' פעמים ל‫' | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{30\times30=\left(3\sdot3\right)\sdot100=9\sdot100=900}}</math> | + | :We take their analogous from the corresponding units: since 30 is 3 tens, their analogous is 3 times 3 and as 3 times 3 is 9, so 30 times 30 is 9 hundred. |
| − | |style="text-align:right;"|ניקח דמיונם מן האחדים הדומים להם ובעבור היות ל'<ref>של' הם‫ MS Cambridge :היות ל‫'</ref> ג' | + | :<math>\scriptstyle{\color{blue}{30\times30=\left(3\sdot3\right)\sdot100=9\sdot100=900}}</math> |
| + | |style="text-align:right;"|ניקח דמיונם מן האחדים הדומים להם ובעבור היות ל'<ref>של' הם‫ MS Cambridge :היות ל‫'</ref> ג'‫<ref>ל' ג': MS Ithaca ג' ל'</ref> עשרו' דמיונם הוא ג' פעמי' ג'<ref>ג'פ'ג‫'‫ MS Cambridge :ג' פעמי' ג‫'</ref> וכמו שג' פעמים ג'<ref>שג'פ'ג‫'‫ MS Cambridge :שג' פעמים ג‫'</ref> הם ט' כן ל' פעמים ל' הם ט' מאות | ||
|- | |- | ||
| | | | ||
| − | : | + | *Also 40 times 40. |
| + | :<math>\scriptstyle40\times40</math> | ||
|style="text-align:right;"|וכן מ' פעמים מ‫'<ref>מ'פ'מ‫'‫ MS Cambridge :מ' פעמים מ‫'</ref> | |style="text-align:right;"|וכן מ' פעמים מ‫'<ref>מ'פ'מ‫'‫ MS Cambridge :מ' פעמים מ‫'</ref> | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{40\times40=\left(4\sdot4\right)\sdot100=16\sdot100=1600}}</math> | + | :Their analogous is 4 times [4], which is 16 hundred. |
| − | |style="text-align:right;"|דמיונם הוא ד' פעמים שהם י"ו מאות‫<ref>ד' ד'פ'ד' י"ו וכן מ'פ'מ' י"ו מאות‫ MS Cambridge :ד' ... מאות</ref> | + | :<math>\scriptstyle{\color{blue}{40\times40=\left(4\sdot4\right)\sdot100=16\sdot100=1600}}</math> |
| + | |style="text-align:right;"|דמיונם הוא ד' פעמים שהם י"ו‫<ref>י"ו: MS Ithaca: י"ו וכן מ' פעמי' מ' הם י"ו</ref> מאות‫<ref>ד' ד'פ'ד' י"ו וכן מ'פ'מ' י"ו מאות‫ MS Cambridge :ד' ... מאות</ref> | ||
|- | |- | ||
| | | | ||
| − | : | + | *Also 30 times 40. |
| + | :<math>\scriptstyle30\times40</math> | ||
|style="text-align:right;"|וכן ל' פעמים מ‫'<ref>ל'פ'מ‫'‫ MS Cambridge :ל' פעמים מ‫'</ref> | |style="text-align:right;"|וכן ל' פעמים מ‫'<ref>ל'פ'מ‫'‫ MS Cambridge :ל' פעמים מ‫'</ref> | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{30\times40=\left(3\sdot4\right)\sdot100=12\sdot100=1200}}</math> | + | :Their analogous is 3 times 4, which is 12. So, 30 times 40 is 12 hundred. |
| − | |style="text-align:right;"|דמיונם הוא ג' פעמים ד'<ref>ג'פ'ד‫'‫ MS Cambridge :הוא ... ד‫'</ref> שהם י"ב וכן ל' פע' מ'<ref>ל'פ'מ‫'‫ MS Cambridge :ל' פע' מ‫'</ref> הם י"ב מאות | + | :<math>\scriptstyle{\color{blue}{30\times40=\left(3\sdot4\right)\sdot100=12\sdot100=1200}}</math> |
| + | |style="text-align:right;"|דמיונם הוא ג' פעמים ד'<ref>ג'פ'ד‫'‫ MS Cambridge :הוא ... ד‫'</ref> שהם י"ב וכן ל' פע' מ'<ref>ל'פ'מ‫'‫ MS Cambridge :ל' פע' מ‫'</ref> הם י"ב מאות | ||
|- | |- | ||
| − | |The | + | |The same for all. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכן כלם |
|- | |- | ||
| − | |The rule for multiplication of | + | |The rule for this is that when we multiply units by tens, we take their analogous from the multiplication of the units by the units and the result is tens. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והכלל המסור לזה הוא כשנרבה אחדים עם עשרות נקח דמיונם מרבוי האחדים עם האחדים ויהיו העולה עשרות |
|- | |- | ||
| + | |If we multiply tens by tens the result is hundreds. | ||
| + | |style="text-align:right;"|ואם נרבה עשרות עם עשרות יהיה העולה מאות‫<ref>ואם נרבה עשרות עם עשרות... מאות: MS Ithaca om.</ref> | ||
| + | |- | ||
| + | !<span style=color:green>Tens by Hundreds</span> | ||
| | | | ||
| − | + | |- | |
| − | :<math>\scriptstyle\left(a\sdot10\right)\times\left(100\sdot b\right)</math> | + | |If we multiply tens by hundreds, the result is thousands. |
| − | |style="text-align:right;"|ואם נרבה עשרות עם מאות יהיה<ref>יהיה: MS Cambridge יהיו</ref> העולה אלפים | + | :<math>\scriptstyle{\color{OliveGreen}{\left(a\sdot10\right)\times\left(100\sdot b\right)}}</math> |
| + | |style="text-align:right;"|ואם נרבה עשרות‫<ref>עשרות: MS Ithaca עשרי'</ref>עם מאות יהיה<ref>יהיה: MS Cambridge יהיו</ref> העולה אלפים | ||
|- | |- | ||
| | | | ||
| − | : | + | *As 20 times 2 hundreds. |
| + | :<math>\scriptstyle20\times200</math> | ||
|style="text-align:right;"|כגון כ' פעמים ב' מאות‫<ref>ב'פ'ר‫'‫ MS Cambridge :כ' פעמים ב' מאות</ref> | |style="text-align:right;"|כגון כ' פעמים ב' מאות‫<ref>ב'פ'ר‫'‫ MS Cambridge :כ' פעמים ב' מאות</ref> | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{20\times200=\left(2\sdot2\right)\sdot1000=4\sdot1000=4000}}</math> | + | :Their analogous is 2 times 2, which is 4. So, it is 4 thousand. |
| − | |style="text-align:right;"|דמיונם ב' פעמים ב'<ref>ב' | + | :<math>\scriptstyle{\color{blue}{20\times200=\left(2\sdot2\right)\sdot1000=4\sdot1000=4000}}</math> |
| + | |style="text-align:right;"|דמיונם שהם ב' פעמים ב'<ref>ב': MS Ithaca om.</ref> שהם ד' והם<ref>כן ב'פ'ר‫'‫ MS Cambridge :והם</ref> ד' אלפים | ||
|- | |- | ||
| | | | ||
| − | : | + | *Also 50 times 500. |
| + | :<math>\scriptstyle50\times500</math> | ||
|style="text-align:right;"|וכן נ' פעמי' ת"ק | |style="text-align:right;"|וכן נ' פעמי' ת"ק | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{50\times500=\left(5\sdot5\right)\sdot1000=25\sdot1000=25000}}</math> | + | :Their analogous is 5 times 5, which is 25. So, it is 25 thousand. |
| − | |style="text-align:right;"|דמיונם ה' פעמי' ה'<ref>ה'פ'ה‫'‫ MS Cambridge :ה' פעמי' ה‫'</ref> שהם<ref>שהם: MS Cambridge יהיו</ref> כ"ה והם<ref>והם: MS Cambridge כן נ' פ' ת"ק יהיו</ref> כ"ה אלפים | + | :<math>\scriptstyle{\color{blue}{50\times500=\left(5\sdot5\right)\sdot1000=25\sdot1000=25000}}</math> |
| + | |style="text-align:right;"|דמיונם ה' פעמי' ה'<ref>ה'פ'ה‫'‫ MS Cambridge :ה' פעמי' ה‫'</ref> שהם<ref>שהם: MS Cambridge יהיו</ref> כ"ה והם<ref>והם: MS Cambridge כן נ' פ' ת"ק יהיו</ref> כ"ה אלפים | ||
|- | |- | ||
| + | |The same for all. | ||
| + | |style="text-align:right;"|וכן כלם | ||
| + | |- | ||
| + | !<span style=color:green>Hundreds by Hundreds</span> | ||
| | | | ||
| − | + | |- | |
| − | :<math>\scriptstyle\left(a\sdot100\right)\times\left(100\sdot b\right)</math> | + | |If we multiply hundreds by hundreds the result is tens of thousands. |
| + | :<math>\scriptstyle{\color{OliveGreen}{\left(a\sdot100\right)\times\left(100\sdot b\right)}}</math> | ||
|style="text-align:right;"|ואם נרבה מאות עם מאות יהיה העולה עשרות מאלפים | |style="text-align:right;"|ואם נרבה מאות עם מאות יהיה העולה עשרות מאלפים | ||
|- | |- | ||
| | | | ||
| − | : | + | *As 200 times 200. |
| + | :<math>\scriptstyle200\times200</math> | ||
|style="text-align:right;"|כגון ר' פעמים ר‫'‫<ref>ר'פ'ר‫'‫ MS Cambridge :ר' פעמים ר‫'</ref> | |style="text-align:right;"|כגון ר' פעמים ר‫'‫<ref>ר'פ'ר‫'‫ MS Cambridge :ר' פעמים ר‫'</ref> | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{200\times200=\left(2\sdot2\right)\sdot10000=4\sdot10000=40000}}</math> | + | :Their analogous is 2 times 2, which is 4. So, it is 4 tens of thousands. |
| − | |style="text-align:right;"|דמיונם הם<ref>הם: MS Cambridge om.</ref> ב' פעמים ב'<ref>ב'פ'ב‫'‫ MS Cambridge :ב' פעמים ב‫'</ref> שהם | + | :<math>\scriptstyle{\color{blue}{200\times200=\left(2\sdot2\right)\sdot10000=4\sdot10000=40000}}</math> |
| + | |style="text-align:right;"|דמיונם הם<ref>הם: MS Cambridge om.</ref> ב' פעמים ב'‫<ref>ב'פ'ב‫'‫ MS Cambridge :ב' פעמים ב‫'</ref> שהם<ref>שהם: MS Cambridge כגון ר'פ'ר' יהיו</ref> ד' ‫<ref>32r</ref>והם ד' עשרות מאלפים‫<ref>מאלפים: MS Cambridge מאלפים והם מ' אלפים</ref> | ||
|- | |- | ||
| | | | ||
| − | : | + | *Also 500 times 500. |
| + | :<math>\scriptstyle500\times500</math> | ||
|style="text-align:right;"|וכן ת"ק פעמים ת"ק | |style="text-align:right;"|וכן ת"ק פעמים ת"ק | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{500\times500=\left(5\sdot5\right)\sdot10000=25\sdot10000=250000}}</math> | + | :Their analogous is 5 times 5, which is 25. So, it is 25 tens of thousands. |
| − | |style="text-align:right;"|דמיונם ה' פעמים ה'<ref>ה'פ'ה‫'‫ MS Cambridge :ה' פעמים ה‫'</ref> שהם כ"ה והם<ref>והם: MS Cambridge ויהיו ת"ק פ' ת"ק</ref> כ"ה עשרות מאלפים | + | :<math>\scriptstyle{\color{blue}{500\times500=\left(5\sdot5\right)\sdot10000=25\sdot10000=250000}}</math> |
| − | | | + | |style="text-align:right;"|דמיונם ה' פעמים ה'<ref>ה'פ'ה‫'‫ MS Cambridge :ה' פעמים ה‫'</ref> שהם כ"ה והם<ref>והם: MS Cambridge ויהיו ת"ק פ' ת"ק</ref> כ"ה עשרות מאלפים |
| − | + | |- | |
| + | |The same for all. | ||
| + | |style="text-align:right;"|וכן כלם | ||
|- | |- | ||
| | | | ||
| − | == The Decimal Ranks == | + | == <span style=color:green>The Decimal Ranks</span>‫<ref>MS Ithaca om.</ref> == |
| − | + | | | |
| − | + | |- | |
| + | |The ranks of numbers one above the other: | ||
| + | |style="text-align:right;"|<big>מדרגות</big> המספרים זו למעלה מזו | ||
|- | |- | ||
| | | | ||
| − | * | + | *The units are in the first rank. |
|style="text-align:right;"|האחדים הם במדרגה הראשונה | |style="text-align:right;"|האחדים הם במדרגה הראשונה | ||
|- | |- | ||
| | | | ||
| − | * | + | *The tens are in the second rank. |
|style="text-align:right;"|העשרות הם במדרגה השנית | |style="text-align:right;"|העשרות הם במדרגה השנית | ||
|- | |- | ||
| | | | ||
| − | * | + | *The hundreds are in the third rank. |
|style="text-align:right;"|המאות הם במדרגה השלישית | |style="text-align:right;"|המאות הם במדרגה השלישית | ||
|- | |- | ||
| | | | ||
| − | * | + | *The thousands are in the fourth rank. |
|style="text-align:right;"|האלפים הם במדרגה הרביעית | |style="text-align:right;"|האלפים הם במדרגה הרביעית | ||
|- | |- | ||
| | | | ||
| − | * | + | *The tens of thousands are in the fifth rank. |
|style="text-align:right;"|העשרות מהאלפים הם במדרגה החמישית | |style="text-align:right;"|העשרות מהאלפים הם במדרגה החמישית | ||
|- | |- | ||
| | | | ||
| − | * | + | *The hundreds of Thousands are in the sixth rank. |
|style="text-align:right;"|המאות מהאלפים הם במדרגה השישית | |style="text-align:right;"|המאות מהאלפים הם במדרגה השישית | ||
| + | |- | ||
| + | |So they rise from rank to rank. | ||
| + | |style="text-align:right;"|וכן עולים ממדרגה למדרגה | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | *If we want to multiply 600 by 4 thousand. |
| + | :<math>\scriptstyle600\times4000</math> | ||
| + | |style="text-align:right;"|<big>ואם</big> נרצה לרבות‫<ref>ואם נרצה לרבות: MS Cambridge אם נרבה</ref> ת"ר‫<ref>ת"ר: MS Cambridge ת"ר <s>אלפים</s></ref> בד' אלפים | ||
|- | |- | ||
| | | | ||
| − | : | + | :Their analogous is 6 times 4, which is 24. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{600\times4000=\left(6\sdot4\right)\sdot100000=24\sdot100000}}</math> |
| + | |style="text-align:right;"|דמיונם ו'פ'ד' שהם‫<ref>שהם: MS Cambridge הם</ref> כ"ד | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{ | + | :If you want to know what [is the rank of] this 24, take the [positional value of] the rank of hundreds, which is three, and count 3 ranks from the thousands. The rank you will reach is the rank of 24 that you have kept and it is the rank of hundreds of thousands. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{OliveGreen}{\left(a\sdot10^n\right)\times\left(b\sdot10^m\right)=\left(a\sdot b\right)\sdot\left(10^n\sdot10^m\right)}}</math> |
| + | |style="text-align:right;"|ואם תרצה לדעת אלו הכ"ד מה יורו קח מעלת המאות שהוא שלשה ומנה מן האלפים ג' מעלות והמעלה שתגיע אליה היא מעלת כ"ד ששמרת‫<ref>ששמרת: MS Cambridge המשמרת</ref> והיא מעלת מאות האלפים‫<ref>מאות האלפים: MS Cambridge המאות מהאלפים</ref> | ||
|- | |- | ||
| | | | ||
| − | + | :Likewise, if you count 4 ranks, which is the rank of thousands, from the rank of hundreds, you reach also the rank of hundreds of thousands. | |
| − | : | + | |style="text-align:right;"|<big>וכן</big> אם אתה מונה מעלת האלפים והוא ד' ממעלת המאות אתה מגיע גם כן<ref>גם כן: MS Cambridge ג"כ</ref> אל מעלת מאות האלפים |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | : | + | :Know then that the units of the number you have kept, which is 24, is in the rank of hundreds of thousands, and its tens are in the rank of thousands of thousands. So, the 24 you have kept are 2 thousands of thousands and 400 thousand, because its units are four and its tens are 2. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ותדע מכאן כי האחדים מהמספר אשר שמרת והוא כ"ד הוא ממעלת מאות האלפים ועשרותיו הם ממעלת אלפי האלפים<ref>האלפים: MS Cambridge אלפים</ref> יהיו אם כן<ref>אם כן: MS Cambridge א"כ</ref> כ"ד אשר שמרת ב' אלפי אלפים ות' אלף כי אחדיו הם ארבעה ועשרותיו הם ב‫' |
|- | |- | ||
| − | | | + | |Another method: consider [the positional values] of the two ranks and know their [sum]. Subtract 1 from it; the remainder is the rank of the product. |
| − | |style="text-align:right;"|דרך אחרת תחשוב אישי [פי' אחדי'] ב' המעלות ותדע מספרם ותפחות מהם א'<ref>אחד | + | |style="text-align:right;"|<big>דרך</big> אחרת תחשוב אישי [פי' אחדי'] ב' המעלות ותדע מספרם ותפחות מהם א'‫<ref>אחד: MS Cambridge א'</ref> והנשאר יהיה מעלת הנכלל |
|- | |- | ||
| | | | ||
| − | : | + | :As if you take [the positional value of] the rank of hundreds in this example, which is 3, and [the positional value of] the rank of thousands, which is 4; they are 7. Subtract 1 from it; 6 remains and this number is [the positional value of] the rank of hundreds of thousands. |
| + | :<math>\scriptstyle{\color{blue}{\left(3+4\right)-1=7-1=6}}</math> | ||
|style="text-align:right;"|כאלו תקח במשל הזה אוש המאות והוא ג' ואוש האלפים והוא ד' יהיו ז' ותפחות מהם א' וישארו ו' וזה המספר הוא אוש מאות האלפים | |style="text-align:right;"|כאלו תקח במשל הזה אוש המאות והוא ג' ואוש האלפים והוא ד' יהיו ז' ותפחות מהם א' וישארו ו' וזה המספר הוא אוש מאות האלפים | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | == Three Types of Numbers | + | == <span style=color:green>Three Types of Numbers</span>‫<ref>MS Ithaca om.</ref> == |
| − | |||
| − | |||
| − | |||
| | | | ||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |The numbers are [of] three [types]: perfect number, abundant number, deficient number. |
| − | + | |style="text-align:right;"|<big>המספרים</big> הם ג' <big>מספר שלם מספר עודף מספר חסר</big> | |
| − | |style="text-align:right;"|מספר עודף | ||
|- | |- | ||
| − | | | + | |The perfect number, as the number 6, its parts divide it and complete it [= it is equal to the sum of its divisors]; they do not exceed it, nor less than it. Because its parts are a half, a sixth, and a third. If you sum them up, they are six, which is the same as it. |
| − | + | :<math>\scriptstyle{\color{blue}{\left(\frac{1}{6}\sdot6\right)+\left(\frac{1}{3}\sdot6\right)+\left(\frac{1}{2}\sdot6\right)=6}}</math> | |
| − | |style="text-align:right;"|מספר | + | |style="text-align:right;"|מספר שלם הוא כמו מספר ו' אשר חלקיו המונים אותו ממלאים אותו ואינם עודפים עליו ואינם‫<ref>ואינם: MS Cambridge ולא</ref> חסרים ממנו כי חלקיו הם חצי ושתות ושליש ואם תקבצם יהיו ששה כמוהו |
|- | |- | ||
| − | | | + | |The same for the number 28 - its parts are: one part of 28, one part of 14, a seventh, a quarter, and a half. If you sum them up, they are 28, which is the same as it. |
| − | + | |style="text-align:right;"|וכן מספר כ"ח חלקיו הם חלק מכ"ח וחלק מי"ד ושביע ורביע וחצי ואם תקבצם יהיו כ"ח כמוהו | |
| − | |style="text-align:right;"| | ||
| − | |||
|- | |- | ||
| − | | | + | | colspan="2"| |
:<math>\scriptstyle{\color{blue}{\left(\frac{1}{28}\sdot28\right)+\left(\frac{1}{14}\sdot28\right)+\left(\frac{1}{7}\sdot28\right)+\left(\frac{1}{4}\sdot28\right)+\left(\frac{1}{2}\sdot28\right)=28}}</math> | :<math>\scriptstyle{\color{blue}{\left(\frac{1}{28}\sdot28\right)+\left(\frac{1}{14}\sdot28\right)+\left(\frac{1}{7}\sdot28\right)+\left(\frac{1}{4}\sdot28\right)+\left(\frac{1}{2}\sdot28\right)=28}}</math> | ||
| − | |||
|- | |- | ||
| − | | | + | |The abundant number, as 12, whose parts are one part of 12, a sixth, a third, a quarter, and a half. If you sum them up, they are 16. |
| + | |style="text-align:right;"|<big>ומספר עודף</big> הוא י"ב שחלקיו הם חלק מי"ב ושתות ושליש ורביע וחצי ואם תקבצם יהיו כלם י"ו | ||
| + | |- | ||
| + | | colspan="2"| | ||
:<math>\scriptstyle{\color{blue}{\left(\frac{1}{12}\sdot12\right)+\left(\frac{1}{6}\sdot12\right)+\left(\frac{1}{3}\sdot12\right)+\left(\frac{1}{4}\sdot12\right)+\left(\frac{1}{2}\sdot12\right)=16>12}}</math> | :<math>\scriptstyle{\color{blue}{\left(\frac{1}{12}\sdot12\right)+\left(\frac{1}{6}\sdot12\right)+\left(\frac{1}{3}\sdot12\right)+\left(\frac{1}{4}\sdot12\right)+\left(\frac{1}{2}\sdot12\right)=16>12}}</math> | ||
| − | |||
|- | |- | ||
| − | | | + | |The deficient number, as 14, whose parts are one part of 14, its half, and its seventh. If you sum them up, they are ten. |
:<math>\scriptstyle{\color{blue}{\left(\frac{1}{14}\sdot14\right)+\left(\frac{1}{7}\sdot14\right)+\left(\frac{1}{2}\sdot14\right)=10<12}}</math> | :<math>\scriptstyle{\color{blue}{\left(\frac{1}{14}\sdot14\right)+\left(\frac{1}{7}\sdot14\right)+\left(\frac{1}{2}\sdot14\right)=10<12}}</math> | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<big>ומספר חסר</big> כגון מספר י"ד שחלקיו הם חלק מי"ד וחציו ושביעו ואם תקבצם יהיו כלם עשר |
| − | + | |- | |
| − | |||
| − | |- | ||
| | | | ||
| − | == The Ten Numerals == | + | == <span style=color:green>The Ten Numerals</span>‫<ref>MS Ithaca om.</ref> == |
| | | | ||
|- | |- | ||
| − | | | + | |The sages of India made the shapes of their digits like these: |
|style="text-align:right;"|וחכמי הודו עשו צורות אותיותם כמו אלו | |style="text-align:right;"|וחכמי הודו עשו צורות אותיותם כמו אלו | ||
|- | |- | ||
| Line 289: | Line 351: | ||
| | | | ||
| − | == Finding the Square Numbers == | + | == <span style=color:green>The Method of Finding the Square Numbers</span>‫<ref>MS Ithaca om.</ref> == |
| − | + | |style="text-align:right;"|<big>הסדר למצא המספרים המרובעים</big> | |
|- | |- | ||
| − | |<math>\scriptstyle n^2+\left(2n+1\right)=\left(n+1\right)^2</math> | + | |It is that you sum the first square with the odd number that follows it and you will find the second square. |
| + | :<math>\scriptstyle{\color{OliveGreen}{n^2+\left(2n+1\right)=\left(n+1\right)^2}}</math> | ||
|style="text-align:right;"|הוא שתקבץ המרובע הראשון עם המספר הנפרד הבא אחריו ותמצא המרובע השני | |style="text-align:right;"|הוא שתקבץ המרובע הראשון עם המספר הנפרד הבא אחריו ותמצא המרובע השני | ||
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle{\color{blue}{1^2+3=4=2^2}}</math> | + | *As if you sum one, which is the first square, with 3, which is the first of the odd numbers; you find 4, which is the second square, whose root is 2. |
| + | :<math>\scriptstyle{\color{blue}{1^2+3=4=2^2}}</math> | ||
|style="text-align:right;"|כמו אם תקבץ האחד שהוא המרובע הראשון עם הג' שהוא ראש<ref>ראש: MS Cambridge תחלת</ref> הנפרדים<ref>הנפרדים: MS Cambridge הנפרדות</ref> תמצא ד' שהוא המרובע השני אשר צלעו ב‫' | |style="text-align:right;"|כמו אם תקבץ האחד שהוא המרובע הראשון עם הג' שהוא ראש<ref>ראש: MS Cambridge תחלת</ref> הנפרדים<ref>הנפרדים: MS Cambridge הנפרדות</ref> תמצא ד' שהוא המרובע השני אשר צלעו ב‫' | ||
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle{\color{blue}{2^2+5=4+5=9=3^2}}</math> | + | *If you sum 4, which is the second square, with 5, which is the second odd number, they are 9, which is the third square, whose root is 3. |
| + | :<math>\scriptstyle{\color{blue}{2^2+5=4+5=9=3^2}}</math> | ||
|style="text-align:right;"|ואם תקבץ ד' שהוא המרובע השני עם ה' שהוא הנפרד השני יהיו ט' והוא המרובע השלישי אשר צלעו ג‫' | |style="text-align:right;"|ואם תקבץ ד' שהוא המרובע השני עם ה' שהוא הנפרד השני יהיו ט' והוא המרובע השלישי אשר צלעו ג‫' | ||
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle{\color{blue}{3^2+7=9+7=16=4^2}}</math> | + | *If you add the third odd number, which is 7, to 9, which is the third square, you find 16, which is the fourth square, whose root is 4. |
| + | :<math>\scriptstyle{\color{blue}{3^2+7=9+7=16=4^2}}</math> | ||
|style="text-align:right;"|ואם אתה מוסיף על ט' שהוא המרובע השלישי המספר הנפרד השלישי שהוא<ref>שהוא: MS Cambridge והוא</ref> ז' תמצא י"ו שהוא המרובע הרביעי אשר צלעו ד‫' | |style="text-align:right;"|ואם אתה מוסיף על ט' שהוא המרובע השלישי המספר הנפרד השלישי שהוא<ref>שהוא: MS Cambridge והוא</ref> ז' תמצא י"ו שהוא המרובע הרביעי אשר צלעו ד‫' | ||
|- | |- | ||
| − | | | + | |This way you find all of them. |
|style="text-align:right;"|ועל זה הסדר תמצאם כלם | |style="text-align:right;"|ועל זה הסדר תמצאם כלם | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | == Cubic Numbers == | + | == <span style=color:green>Cubic Numbers</span>‫<ref>MS Ithaca om.</ref> == |
| − | |||
| | | | ||
|- | |- | ||
| − | | | + | |Anything whose length, breadth, and depth are equal is called cubic. |
| − | |style="text-align:right;"|כל דבר שארכו ורחבו ועמקו שוים יקרא | + | |style="text-align:right;"|<big>כל</big> דבר שארכו ורחבו ועמקו שוים יקרא מעוקב |
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle{\color{blue}{1^3=1}}</math> | + | *1 is the first cubic number. It is a place that is 1 in length, 1 in width, 1 in height and 1 in depth. |
| + | :<math>\scriptstyle{\color{blue}{1^3=1}}</math> | ||
|style="text-align:right;"|והנה א' הוא המעוקב הראשון והוא מקום שארכו א' ורחבו א' וגבהו א' ועמקו א‫' | |style="text-align:right;"|והנה א' הוא המעוקב הראשון והוא מקום שארכו א' ורחבו א' וגבהו א' ועמקו א‫' | ||
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle{\color{blue}{2^3=8}}</math> | + | *8 is the second cubic number derived from a place whose length, width, and depth are 2. |
| + | :<math>\scriptstyle{\color{blue}{2^3=8}}</math> | ||
|style="text-align:right;"|וח' הוא המעקב השני היוצא ממקום שארכו ורחבו ועמקו ב‫' | |style="text-align:right;"|וח' הוא המעקב השני היוצא ממקום שארכו ורחבו ועמקו ב‫' | ||
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle{\color{blue}{3^3=27}}</math> | + | *27 is the third cubic number derived from a place whose length, width, and depth are 3. |
| + | :<math>\scriptstyle{\color{blue}{3^3=27}}</math> | ||
|style="text-align:right;"|וכ"ז הוא המעוקב השלישי היוצא ממקום שארכו ורחבו ועמקו ג‫' | |style="text-align:right;"|וכ"ז הוא המעוקב השלישי היוצא ממקום שארכו ורחבו ועמקו ג‫' | ||
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle{\color{blue}{4^3=64}}</math> | + | *64 is the fourth cubic number derived from a place whose length, width, and depth are 4. |
| + | :<math>\scriptstyle{\color{blue}{4^3=64}}</math> | ||
|style="text-align:right;"|וס"ד הוא המעקב הרביעי היוצא ממקום שארכו ורחבו ועמקו ד‫' | |style="text-align:right;"|וס"ד הוא המעקב הרביעי היוצא ממקום שארכו ורחבו ועמקו ד‫' | ||
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle{\color{blue}{5^3=125}}</math> | + | *125 is the fifth cubic number derived from a place whose length, width, and depth are 5. |
| + | :<math>\scriptstyle{\color{blue}{5^3=125}}</math> | ||
|style="text-align:right;"|וקכ"ה הוא המעוקב החמשי היוצא ממקום שארכו ורחבו ועמקו ועמקו ה‫' | |style="text-align:right;"|וקכ"ה הוא המעוקב החמשי היוצא ממקום שארכו ורחבו ועמקו ועמקו ה‫' | ||
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle{\color{blue}{6^3=216}}</math> | + | *216 is the sixth cubic number derived from a place whose length, width, and depth are 6. |
| + | :<math>\scriptstyle{\color{blue}{6^3=216}}</math> | ||
|style="text-align:right;"|ורי"ו הוא המעוקב הששי היוצא ממקום שארכו ורחבו ועמקו ו‫' | |style="text-align:right;"|ורי"ו הוא המעוקב הששי היוצא ממקום שארכו ורחבו ועמקו ו‫' | ||
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle{\color{blue}{7^3=343}}</math> | + | *343 is the seventh cubic number derived from a place whose length, width, and depth are 7. |
| + | :<math>\scriptstyle{\color{blue}{7^3=343}}</math> | ||
|style="text-align:right;"|ושמ"ג הוא המעקב השבעי היוצא ממקום שארכו ורחבו ועמקו ז‫' | |style="text-align:right;"|ושמ"ג הוא המעקב השבעי היוצא ממקום שארכו ורחבו ועמקו ז‫' | ||
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle{\color{blue}{8^3=512}}</math> | + | *512 is the eighth cubic number derived from a place whose length, width, and depth are 8. |
| + | :<math>\scriptstyle{\color{blue}{8^3=512}}</math> | ||
|style="text-align:right;"|ותקי"ב הוא המעוקב השמני היוצא ממקום שארכו ורחבו ועמקו ח‫' | |style="text-align:right;"|ותקי"ב הוא המעוקב השמני היוצא ממקום שארכו ורחבו ועמקו ח‫' | ||
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle{\color{blue}{9^3=729}}</math> | + | *729 is the ninth cubic number derived from a place whose length, width, and depth are 9. |
| + | :<math>\scriptstyle{\color{blue}{9^3=729}}</math> | ||
|style="text-align:right;"|ותשכ"ט הוא המעוקב התשעי היוצא ממקום שארכו ורחבו ועמקו ט‫' | |style="text-align:right;"|ותשכ"ט הוא המעוקב התשעי היוצא ממקום שארכו ורחבו ועמקו ט‫' | ||
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle{\color{blue}{10^3=1000}}</math> | + | *One thousand is the tenth cubic number derived from a place whose length, width, and depth are 10. |
| + | :<math>\scriptstyle{\color{blue}{10^3=1000}}</math> | ||
|style="text-align:right;"|ואלף הוא המעוקב העשרי היוצא ממקום שארכו ורחבו ועמקו י‫' | |style="text-align:right;"|ואלף הוא המעוקב העשרי היוצא ממקום שארכו ורחבו ועמקו י‫' | ||
|- | |- | ||
| − | |<math>\scriptstyle\left(2n\right)^3=8\sdot n^3</math> | + | |You see that every cubic number, [when it is] doubled, is 8 times the original [cubic] number. |
| + | :<math>\scriptstyle{\color{OliveGreen}{\left(2n\right)^3=8\sdot n^3}}</math> | ||
|style="text-align:right;"|והנך רואה כי כל מעוקב [זוג] יהיה ח' פעמי' מהמספר הראשון | |style="text-align:right;"|והנך רואה כי כל מעוקב [זוג] יהיה ח' פעמי' מהמספר הראשון | ||
|- | |- | ||
| | | | ||
| + | *Because the cube of 1 is 1. | ||
| + | :<math>\scriptstyle{\color{blue}{1^3=1}}</math> | ||
|style="text-align:right;"|כי כל מעקב א' הוא א‫' | |style="text-align:right;"|כי כל מעקב א' הוא א‫' | ||
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle{\color{blue}{2^3=\left(2\sdot1\right)^3=8=8\sdot1^3}}</math> | + | *The cube of 2, which is double 1, is 8. |
| + | :<math>\scriptstyle{\color{blue}{2^3=\left(2\sdot1\right)^3=8=8\sdot1^3}}</math> | ||
|style="text-align:right;"|ומעקב ב' שהוא כפל א' הוא ח‫' | |style="text-align:right;"|ומעקב ב' שהוא כפל א' הוא ח‫' | ||
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle{\color{blue}{4^3=\left(2\sdot2\right)^3=64=8\sdot2^3}}</math> | + | *The cube of 4, which is double 2, is 64, which is 8 times the cube of 2. |
| + | :<math>\scriptstyle{\color{blue}{4^3=\left(2\sdot2\right)^3=64=8\sdot2^3}}</math> | ||
|style="text-align:right;"|ומעקב ד' שהוא כפל ב' הוא ס"ד שהוא ח' פ' במעקב ב‫' | |style="text-align:right;"|ומעקב ד' שהוא כפל ב' הוא ס"ד שהוא ח' פ' במעקב ב‫' | ||
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle{\color{blue}{6^3=8\sdot3^3}}</math> | + | *Also the cube of 6 is 8 times the cube of 3. |
| − | |style="text-align:right;"|וכן מעקב ו' הוא ח' פ' במעקב ג' | + | :<math>\scriptstyle{\color{blue}{6^3=8\sdot3^3}}</math> |
| − | | | + | |style="text-align:right;"|וכן מעקב ו' הוא ח' פ' במעקב ג‫' |
| − | + | |- | |
| + | |And so on. | ||
| + | |style="text-align:right;"|וכן לעולם | ||
|- | |- | ||
| − | + | !<span style=color:green>Word Problem - Payment Problem</span> | |
| | | | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:digging a hole|612|QJWz}} | + | *{{#annot:digging a hole|612|QJWz}}Therefore, if someone says: dig me a hole 2 in length, 2 in breadth, 2 in depth, the payment should be 40 liṭra, which is 8 times 5 liṭra. |
:<math>\scriptstyle{\color{blue}{2^3\sdot5=8\sdot5=40}}</math> | :<math>\scriptstyle{\color{blue}{2^3\sdot5=8\sdot5=40}}</math> | ||
| − | |style="text-align:right;"|ולכן מי שיאמר חפור לי בור שיהיה ב' ארכו ב' רחבו ב' עמקו יהיה שכרו מ' ליט' שהוא ח' פעמי' ה' ליט'{{#annotend:QJWz}} | + | |style="text-align:right;"|ולכן מי שיאמר חפור לי בור שיהיה ב' ארכו ב' רחבו ב' עמקו יהיה שכרו מ' ליט' שהוא ח' פעמי' ה' ליט‫'{{#annotend:QJWz}} |
|- | |- | ||
| − | | | + | |Because every cubic number, when it is doubled, is 8 times the original [cubic] number. |
|style="text-align:right;"|כי כל מעקב כשיכפול יהיה ח' פעמים מהמספר הראשון | |style="text-align:right;"|כי כל מעקב כשיכפול יהיה ח' פעמים מהמספר הראשון | ||
|- | |- | ||
| | | | ||
| − | == Primary Definitions == | + | == <span style=color:green>Primary Definitions</span>‫<ref>MS Ithaca om.</ref> == |
| − | |||
| | | | ||
|- | |- | ||
| | | | ||
| − | * | + | *<big>The unity</big> is the issue by which every one of the beings in the world is called one. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|האחדות הוא הענין אשר בו קוראים לכל אחד מהנמצאי' בעולם אחד |
|- | |- | ||
| | | | ||
| − | * | + | *<big>The number</big> is the multitude summed from units. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|המספר הוא הרבוי הנקבץ מן האחדים |
|- | |- | ||
| | | | ||
| − | * | + | *<big>The number counted by another number</big> is the product of the counted number multiplied a number of times that equals to the number of the units contained in the other number by which it is counted. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|המספר המנוי במספר אחר הוא מספר הנקבץ מכפל המספר המנוי פעמי' אשר מניינם כמניין האחדים אשר במספר השני אשר הוא נמנה בו |
|- | |- | ||
| | | | ||
| − | * | + | *<big>The square number</big> is the product of a number by the number of the units contained in that number itself. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|המספר המרובע הוא המספר הנקבץ מכפל מספר במספר אחדיו הנמצאי' בו באותו המספר עצמו |
|- | |- | ||
| | | | ||
| − | * | + | *<big>The the original number</big> is the root of the square number. |
| − | |style="text-align:right;"|המספר הראשון הוא | + | |style="text-align:right;"|המספר הראשון הוא גדר המרובע |
|- | |- | ||
| | | | ||
| − | :: | + | ::As the number nine, which is called a square, because it is a product of three times three. |
| + | ::<math>\scriptstyle{\color{blue}{9=3^2}}</math> | ||
|style="text-align:right;"|כגון מספר תשעה נקרא מרובע מפני שהוא נקבץ מכפל השלשה שלשה פעמי‫' | |style="text-align:right;"|כגון מספר תשעה נקרא מרובע מפני שהוא נקבץ מכפל השלשה שלשה פעמי‫' | ||
|- | |- | ||
| | | | ||
| − | :: | + | ::The number three is called the root of the square. |
| + | ::<math>\scriptstyle{\color{blue}{3=\sqrt{9}}}</math> | ||
|style="text-align:right;"|ומספר השלשה נקרא גדר המרובע | |style="text-align:right;"|ומספר השלשה נקרא גדר המרובע | ||
|- | |- | ||
| | | | ||
| − | * | + | *<big>The cubic number</big> is an equilateral body, whose length, breadth and depth are equal and this number is the product of a square number by its root. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והמספר מעקב הוא הגוף השוה שארכו ורחבו ועמקו שוים והמספר הזה הוא המספר הנקבץ מכפל מספר המרובע כמנין גדרו |
|- | |- | ||
| | | | ||
| − | :: | + | ::As the number 9, which is a square; if you multiply it by 3, which is its root, the product is 27 and this number is called a cubic number. |
| + | ::<math>\scriptstyle{\color{blue}{27=9\sdot3=3^2\sdot3}}</math> | ||
|style="text-align:right;"|כגון מספר ט' שהוא 9 מרובע אם אתה כופל אותו במניין ג' שהוא גדרו יהיה הנקבץ מזה כ"ז והמספר הזה הוא הנקרא מספר מעוקב | |style="text-align:right;"|כגון מספר ט' שהוא 9 מרובע אם אתה כופל אותו במניין ג' שהוא גדרו יהיה הנקבץ מזה כ"ז והמספר הזה הוא הנקרא מספר מעוקב | ||
|- | |- | ||
| | | | ||
| − | * | + | *Relating a number to another number, or a shape to another shape - ? |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|הקשת מניין אל מניין או תמונה לתמונה או ערך אחר כן השני |
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | == Multiplication == | + | == <span style=color:green>Multiplication</span> == |
| | | | ||
|- | |- | ||
| | | | ||
| − | === Units and tens by units and tens === | + | === <span style=color:green>Units and tens by units and tens</span> === |
| − | + | | | |
| − | !style="text-align:right;"|דרך רבוי ב' אותיות כנגד ב' אותיות | + | |- |
| + | !The method of multiplying two digits by two digits | ||
| + | |style="text-align:right;"|<big>דרך רבוי ב' אותיות כנגד ב' אותיות</big>‫<ref>דרך...ב' אותיות: MS Ithaca om.</ref> | ||
|- | |- | ||
| − | | | + | |When we wish to multiply two digits by two digits: |
| − | |style="text-align:right;"|כשנרצה<ref>כשנרצה: MS Cambridge כשתרצה</ref> לרבות ב' אותיות כנגד ב' אותיות | + | |style="text-align:right;"|<big>כשנרצה</big>‫<ref>כשנרצה: MS Cambridge כשתרצה</ref> לרבות ב' אותיות כנגד ב' אותיות |
|- | |- | ||
| − | | | + | |We multiply them three times one after another: first, we multiply the units by the units; then we multiply the units by the tens; and then we multiply the tens by the tens. |
|style="text-align:right;"|נרבה אותם ג' פעמי' זה אחר זה<br> | |style="text-align:right;"|נרבה אותם ג' פעמי' זה אחר זה<br> | ||
בראשונה נרבה האחדים עם האחדים<br> | בראשונה נרבה האחדים עם האחדים<br> | ||
| − | ואחרי כן<ref>ואחרי כן: MS Cambridge ואח"כ</ref> נרבה האחדים עם העשרות<br> | + | ואחרי כן<ref>ואחרי כן: MS Cambridge ואח"כ</ref> נרבה האחדים עם <s>ב</s> העשרות<br> |
ואחרי כן<ref>ואחרי כן: MS Cambridge ואח"כ</ref> נרבה העשרות עם העשרות | ואחרי כן<ref>ואחרי כן: MS Cambridge ואח"כ</ref> נרבה העשרות עם העשרות | ||
|- | |- | ||
| − | | | + | |Here is the example of the [ordered] multiplications: |
|style="text-align:right;"|והנה דמיון הריבוי הקודם והמתאחר | |style="text-align:right;"|והנה דמיון הריבוי הקודם והמתאחר | ||
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle22\times22</math> | + | *We wish to multiply 22 times 22. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle22\times22</math> |
| + | |style="text-align:right;"|<big>רצינו</big> לרבות כ"ב פעמים כ"ב | ||
|- | |- | ||
| | | | ||
| Line 502: | Line 585: | ||
|- | |- | ||
| | | | ||
| + | :First, we multiply the units by each other and say: 2 times 2 is 4. We write 4 above the units on the right. | ||
| + | |style="text-align:right;"|נרבה בתחילת האחדים זה עם זה ונאמר ב' פעמים ב' הם ד' ונכתוב ד' למעלה מן האחדים מצד ימין | ||
| + | |- | ||
| + | | | ||
| + | :Then, we multiply the units by the tens and say: 2 times [2] and 2 times [2] is 8. We write 8. | ||
| + | |style="text-align:right;"|אחרי כן נרבה האחדים עם העשרות ונאמר ב' פעמי' ד' וב' פעמים ד' הרי ח' ונכתוב ח‫' | ||
| + | |- | ||
| + | | | ||
| + | :Then, we multiply the tens by each other and say: 2 times 2 is 4. We write 4. | ||
| + | |style="text-align:right;"|אחרי כן נרבה העשרות זו עם זו ונאמר ב' פעמים ב' ד' ונכתוב ד‫' | ||
| + | |- | ||
| + | | | ||
| + | :We receive that 22 times 22 is 484. | ||
| + | |style="text-align:right;"|יצא לנו כי כ"ב פעמים כ"ב הם תפ"ד | ||
| + | |- | ||
| + | | colspan="2"| | ||
:{| | :{| | ||
|- | |- | ||
| Line 511: | Line 610: | ||
|2<span style="color:red">2</span>||<span style="color:red">22</span>||<span style="color:red">2</span>2|| 22 | |2<span style="color:red">2</span>||<span style="color:red">22</span>||<span style="color:red">2</span>2|| 22 | ||
|} | |} | ||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle44\times44</math> | + | *Also if we wish to multiply 44 times 44. |
| + | :<math>\scriptstyle44\times44</math> | ||
|style="text-align:right;"|וכן אם רצינו לרבות מ"ד פעמים מ"ד | |style="text-align:right;"|וכן אם רצינו לרבות מ"ד פעמים מ"ד | ||
|- | |- | ||
| Line 537: | Line 633: | ||
|- | |- | ||
| | | | ||
| + | :First, we multiply the units by each other and say: 4 times 4 is 16. We write 6 and keep the ten, which is 1. | ||
| + | |style="text-align:right;"|נרבה האחדים זה עם זה בתחילה ונאמר ד' פעמי' ד' הם י"ו ונכתוב ו' ונחזיק‫<ref>ונחזיק: MS Ithaca ונכתוב</ref> בידינו העשרון שהוא א‫' | ||
| + | |- | ||
| + | | | ||
| + | :Then, we multiply the units by the tens and say: 4 times 4 is 16 and 4 times 4 is 16; it is 32. Add to it the 1 you have kept; it is 33. We write 3 and keep 3. | ||
| + | |style="text-align:right;"|אחר כן נרבה האחדים עם העשרות ונאמר ד' פעמי' ד' י"ו וד' פעמים ד' י"ו הרי ל"ב וצרף עמהם הא' שהחזקת בידך הרי ל"ג ונכתוב ג' ונחזיק בידינו ג‫' | ||
| + | |- | ||
| + | | | ||
| + | :Then, we multiply the tens by the tens and say: 4 times 4 is 16; with the 3 we have kept, it is 19. We write 19. | ||
| + | |style="text-align:right;"|אחרי כן נרבה העשרות עם העשרות נאמר ד' פעמים ד' י"ו ועם הג' שהחזקנו בידינו הרי י"ט ונכתוב י"ט | ||
| + | |- | ||
| + | | | ||
| + | :The result is that 44 times 44 is 1936. | ||
| + | |style="text-align:right;"|היוצא מזה כי מ"ד פעמים מ"ד הם אלף ות"תקל"ו | ||
| + | |- | ||
| + | | colspan="2"| | ||
:{| | :{| | ||
|- | |- | ||
| Line 545: | Line 657: | ||
|2<span style="color:red">4</span>||<span style="color:red">44</span>||<span style="color:red>4</span>4||  44 | |2<span style="color:red">4</span>||<span style="color:red">44</span>||<span style="color:red>4</span>4||  44 | ||
|} | |} | ||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | *<math>\ | + | *Also if we wish to multiply 20 times 20. |
| + | :<math>\scriptstyle20\times20</math> | ||
|style="text-align:right;"|וכן אם רצינו לרבות כף פעמים כף | |style="text-align:right;"|וכן אם רצינו לרבות כף פעמים כף | ||
|- | |- | ||
| Line 588: | Line 697: | ||
|- | |- | ||
| | | | ||
| + | :We say: zero times zero is zero. We write it. | ||
| + | |style="text-align:right;"|נאמר ציפרא פעם ציפרא ציפרא ונכתבנה | ||
| + | |- | ||
| + | | | ||
| + | :Then, we say: zero times 2 and 2 times zero is zero. We write it. | ||
| + | |style="text-align:right;"|אחר כך נאמר ציפרא פעמים פעם ב' וב' פעמים ציפרא ציפרא ונכתבנה | ||
| + | |- | ||
| + | | | ||
| + | :Then, we sa: 2 times 2 is 4. We write it. | ||
| + | |style="text-align:right;"|ואחר כך נאמ' ב' פעמים ב' הם ד' ונכתבם | ||
| + | |- | ||
| + | | | ||
| + | :We receive that 20 times 20 is four hundred. | ||
| + | |style="text-align:right;"|יצא לנו כי כ פעמים כ הם ארבע מאות | ||
| + | |- | ||
| + | | colspan="2"| | ||
:{| | :{| | ||
|- | |- | ||
| Line 597: | Line 722: | ||
|2<span style="color:red">0</span>||<span style="color:red">20</span>||<span style="color:red">2</span>0|| 20 | |2<span style="color:red">0</span>||<span style="color:red">20</span>||<span style="color:red">2</span>0|| 20 | ||
|} | |} | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | === Units by units and tens === | + | === <span style=color:green>Units by units and tens</span> === |
| − | + | | | |
| − | |style="text-align:right;"|ואם רצינו לרבות אות אחד שנגד ב אותיות | + | |- |
| + | |If we want to multiply one digit by two digits. | ||
| + | |style="text-align:right;"|<big>ואם רצינו</big> לרבות אות אחד שנגד ב אותיות | ||
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle7\times45</math> | + | *For example, when we wish to know [how much is] 7 times 45, as you see in the diagram: |
| + | :<math>\scriptstyle7\times45</math> | ||
|style="text-align:right;"|כגון שרצינו לדעת ז' פעמים מ"ה כמו שתראה בצורה | |style="text-align:right;"|כגון שרצינו לדעת ז' פעמים מ"ה כמו שתראה בצורה | ||
|- | |- | ||
| Line 643: | Line 765: | ||
|} | |} | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"|נרבה אותם עם הציפרא כדי | + | :We multiply them [by adding] a zero, so that they will be two digits by two digits and this will be done easily. |
| + | :<math>\scriptstyle{\color{blue}{07\times45}}</math> | ||
| + | |style="text-align:right;"|נרבה אותם עם הציפרא כדי שיהיו ב' אותיות כנגד ב' אותיות ויעשה אותם בנקל | ||
| + | |- | ||
| + | | | ||
| + | :We say: 5 times 7 is 35. We write the units, which is 5, and keep the tens that are 3. | ||
| + | |style="text-align:right;"|ונאמר ה' פעמי' ז' ל"ה ונכתוב האחדים שהם ה' ונחזיק בידינו העשרות שהם ג‫' | ||
| + | |- | ||
| + | | | ||
| + | :Then, we say: zero times 5 is zero and 4 times 7 is 28. Add the 3 that we have kept; it is 31. We write 31, for we have nothing left to multiply. | ||
| + | |style="text-align:right;"|אחר כך נאמר ציפרא פע' ה' ציפרא וד' פעמים ז' כ"ח וצרף עמהם הג' שהחזקנו בידינו יהיו ל"א ונכתוב ל"א כי מעתה לא נשאר לנו לרבות דבר אחר | ||
| + | |- | ||
| + | | | ||
| + | :Because, zero times 4 we have left to multiply is zero; so the result is nothing. | ||
| + | |style="text-align:right;"|כי ציפרא פעם ד' שנשאר לנו לרבות הוא ציפרא ואינם עולים לכלום | ||
|- | |- | ||
| | | | ||
| + | :We receive that 7 times 45 is 315. | ||
| + | |style="text-align:right;"|יצא לנו כי ז' פעמים מ"ה הם שט"ו | ||
| + | |- | ||
| + | | colspan="2"| | ||
:{| | :{| | ||
|- | |- | ||
| Line 655: | Line 795: | ||
|4<span style="color:red">5</span>||<span style="color:red">45</span>||<span style="color:red>4</span>5|| 45 | |4<span style="color:red">5</span>||<span style="color:red">45</span>||<span style="color:red>4</span>5|| 45 | ||
|} | |} | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | === Check === | + | ==== <span style=color:green>Check</span> ==== |
| − | + | | | |
| + | |- | ||
| + | |If we want to check if the number we received is correct: | ||
|style="text-align:right;"|ואם נרצה לבחון אם החשבון שיצא לנו הוא אמתי | |style="text-align:right;"|ואם נרצה לבחון אם החשבון שיצא לנו הוא אמתי | ||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle22\times22</math> | + | *We do this way with the calculation of 22 times 22: |
| + | :<math>\scriptstyle22\times22</math> | ||
|style="text-align:right;"|נעשה על זה הדרך בזה החשבון שהוא כ"ב פעמים כ"ב | |style="text-align:right;"|נעשה על זה הדרך בזה החשבון שהוא כ"ב פעמים כ"ב | ||
|- | |- | ||
| Line 692: | Line 826: | ||
|- | |- | ||
| | | | ||
| − | :: | + | :We sum up the upper digits and say: 2 and 2 is 4. We write it next to the two bottom digits. |
| − | |style="text-align:right;"|נקבץ הב' אותיות העליונות ונאמר ב' וב' הם ד' ונכתוב אותם אצל שתי האותיות התחתונות | + | |style="text-align:right;"|נקבץ הב' אותיות העליונות ונאמר ב' וב' הם ד' ונכתוב אותם אצל שתי האותיות התחתונות |
| − | אחרי כן נרבה אלו שתי | + | |- |
| − | וכמו שנשארו בידינו כן צריך שישארו בידינו ז' מן ת'פ'ד' שעולה החשבון אחר שנשליך מהם הט‫' | + | | |
| + | :Then, we multiply the two digits we wrote one above the other and say: 4 times [4] is 16. | ||
| + | |style="text-align:right;"|אחרי כן נרבה אלו שתי אותיות שכתבנו זו עם זו ונאמר ד' פעמי' י"ו | ||
| + | |- | ||
| + | | | ||
| + | :We cast out the 9 from it; 7 remains. We write 7 between the two mentioned digits. | ||
| + | |style="text-align:right;"|נשליך מהם הט' ישארו ז' ונכתוב ז' בתוך שתי האותיות הנזכרות | ||
| + | |- | ||
| + | | | ||
| + | :As the 7 we have left, so we should have 7 left from 484, which is the result of the calculation, after we cast out the 9 from it. | ||
| + | |style="text-align:right;"|וכמו שנשארו בידינו ז' כן צריך שישארו בידינו ז' מן ת'פ'ד' שעולה החשבון אחר שנשליך מהם הט‫' | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | :<math>\scriptstyle{\color{blue}{\begin{cases}\scriptstyle2+2=4\\\scriptstyle2+2=4\end{cases}\longrightarrow 4\times4=16\longrightarrow16\equiv_97}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :If we sum up 4, 8, and 4, which are the digits indicating 484; it is 16. | ||
| + | :<math>\scriptstyle{\color{blue}{4+8+4=16\longrightarrow16\equiv_97}}</math> | ||
| + | |style="text-align:right;"|והנה אם נקבץ ד' וח' וד' שהם האותיות המורות ת'פ'ד' יהיו י"ו | ||
|- | |- | ||
| | | | ||
| − | : | + | :If we cast out 9 from it, 7 remains. So, the calculation is correct. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואם נשליך מהם הט' ישארו <s>ד</s> ז' והוא חשבון אמתי |
| − | ואם נשליך מהם הט' ישארו ז' והוא חשבון אמתי | ||
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle44\times44</math> | + | *Also in the second calculation we did, which is 44 times 44. |
| + | :<math>\scriptstyle44\times44</math> | ||
|style="text-align:right;"|וכן בחשבון השני שעשינו שהם מ"ד פעמים מ"ד | |style="text-align:right;"|וכן בחשבון השני שעשינו שהם מ"ד פעמים מ"ד | ||
|- | |- | ||
| Line 723: | Line 875: | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\begin{cases}\scriptstyle4+4=8\\\scriptstyle4+4=8\end{cases}\longrightarrow 8\times8=64\longrightarrow64\equiv_91}}</math> | + | :We sum up the upper digits and say: 4 and 4 is 8. We write it. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|נקבץ ‫<ref>32v</ref>האותיות העליונות ונאמר ד' וד' הם ח' ונכתבם |
| − | + | |- | |
| + | | | ||
| + | :We multiply them by each other and say: 8 times 8 is 64. | ||
| + | |style="text-align:right;"|ונרבה אותם זו עם זו ונאמר ח' פע' ח' הם ס"ד | ||
| + | |- | ||
| + | | | ||
| + | :Cast out the nines from it; 1 remains. | ||
| + | |style="text-align:right;"|השליכם מהם ט' ט' ישאר מהם א‫' | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | :<math>\scriptstyle{\color{blue}{\begin{cases}\scriptstyle4+4=8\\\scriptstyle4+4=8\end{cases}\longrightarrow 8\times8=64\longrightarrow64\equiv_91}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :The same should remain from 1936, whose digits are 1, 9, 3, 6. | ||
| + | |style="text-align:right;"|וכן צריך להשאר מאלף ת"תק'ל'ו' שסימנם הוא א' וט' וג' וו‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | :Sum them up; it is 19. Cast out the nines from it; the remainder is 1. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואם <s>תכ</s> תקבצם יהיו י"ט ותשליך אותם ט'ט' יהיה הנשאר א‫' |
| − | + | |- | |
| + | | colspan="2"| | ||
| + | :<math>\scriptstyle{\color{blue}{1+9+3+6\longrightarrow19\equiv_91}}</math> | ||
|- | |- | ||
| | | | ||
| − | === Units, tens, and hundreds by units, tens, and hundreds === | + | === <span style=color:green>Units, tens, and hundreds by units, tens, and hundreds</span> === |
| − | + | | | |
| − | |style="text-align:right;"|ואם נרצה לרבות ג' אותיות כנגד ג' אותיות | + | |- |
| + | |If we want to multiply three digits by three digits. | ||
| + | |style="text-align:right;"|<big>ואם נרצה</big> לרבות ג' אותיות כנגד ג' אותיות | ||
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle222\times222</math> | + | *As when we wish to know [the product of] 222 times 222. |
| + | :<math>\scriptstyle222\times222</math> | ||
|style="text-align:right;"|כגון שרצינו לדעת רכ"ב פעמים רכ"ב פעמים | |style="text-align:right;"|כגון שרצינו לדעת רכ"ב פעמים רכ"ב פעמים | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"|נרבה אותם ה' פעמים | + | :We multiply them five times [= i.e. five phases of multiplication, five ranks in the final result of multiplication] and this is the method of multiplication: |
| + | |style="text-align:right;"|נרבה אותם ה' פעמים וזהו דרך ריבויים | ||
| + | |- | ||
| + | | | ||
| + | :We write them 5 times, so that the first, second, third, fourth, and fifth multiplications will be clear to the eye. | ||
| + | |style="text-align:right;"|ונכתוב אותם ה' פעמים כדי שיתבאר למראית העין הרבוי הראשון והשני והשלישי והרביעי וחמשי | ||
|- | |- | ||
| − | | | + | | |
| − | | | + | | |
{|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
|- | |- | ||
| Line 762: | Line 938: | ||
|- | |- | ||
| | | | ||
| + | :If we want to multiply 222 times 222. | ||
|style="text-align:right;"|ואם נרצה לרבות רכ"ב פעמים רכ"ב | |style="text-align:right;"|ואם נרצה לרבות רכ"ב פעמים רכ"ב | ||
| + | |- | ||
| + | | | ||
| + | | | ||
{|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
|- | |- | ||
| Line 778: | Line 958: | ||
|- | |- | ||
| | | | ||
| + | :We start to multiply the units and say: 2 times 2 is 4. We write it. | ||
| + | |style="text-align:right;"|נתחיל לרבות האחדים ונאמר ב' פעמים ב' הם ד' ונכתבם | ||
| + | |- | ||
| + | | | ||
| + | :Then, we multiply the units by the tens and say: 2 times 2 is 4 and 2 times 2 is 4; so, it is 8. We write it. | ||
| + | |style="text-align:right;"|אחר זה נרבה האחדי' עם העשרות ונאמר ב' פעמי' ב' הם ד' וב' פעמים ב' הם ד' הרי ח' ונכתבם‫<ref>ונכתבם: MS Ithaca om.</ref> | ||
| + | |- | ||
| + | | colspan="2"| | ||
:{| | :{| | ||
|- | |- | ||
| Line 786: | Line 974: | ||
|22<span style="color:red">2</span>||2<span style="color:red">22</span>||<span style="color:red>222</span> | |22<span style="color:red">2</span>||2<span style="color:red">22</span>||<span style="color:red>222</span> | ||
|} | |} | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| + | :Then, we multiply all three together and say: 2 times 2 is 4; 2 times 2 is 4; so it is 8; and 2 times 2 is 4; so it is 12. We write 2 and keep 1. | ||
| + | |style="text-align:right;"|אחר זה נרבה שלשתם ביחד ונאמר ב' פעמים ב' ד' וב' פעמים ב' ד' הרי ח'‫<ref>אחר זה נרבה... הרי ח': MS Ithaca om.</ref> וב'פעמים ב' ד' הרי י"ב ונכתוב ב' ונחזי' בידינו א‫' | ||
| + | |- | ||
| + | | colspan="2"| | ||
::::{| | ::::{| | ||
|- | |- | ||
| Line 798: | Line 988: | ||
|<span style="color:red">22</span>2 | |<span style="color:red">22</span>2 | ||
|} | |} | ||
| − | |||
|- | |- | ||
| | | | ||
| + | :We multiply the tens by the hundreds and say: 2 times 2 is 4 and 2 times 2 is 4; so it is 8; with the 1 we have kept, it is 9. We write it. | ||
| + | |style="text-align:right;"|אחר זה נרבה העשרות עם המאות ונאמר ב' פעמים ב' ד' וב' פעמי' ב' ד' הרי ח' וא' שהחזקנו בידינו הרי ט' ונכתבם | ||
| + | |- | ||
| + | | | ||
| + | :We multiply the hundreds by the hundreds and say: 2 times 2 is 4. We write it. | ||
| + | |style="text-align:right;"|אחר זה נרבה המאות עם המאות ונאמר ב' פעמי' ב' ד' ונכתבם | ||
| + | |- | ||
| + | | | ||
| + | :We receive that 222 times 222 is 49284. | ||
| + | |style="text-align:right;"|יצא לנו כי רכ"ב פעמים רכ"ב הם מ"ט אלפים ורפ"ד | ||
| + | |- | ||
| + | | colspan="2"| | ||
:{| | :{| | ||
|- | |- | ||
| Line 809: | Line 1,010: | ||
| <span style="color:red">2</span>22||  222 | | <span style="color:red">2</span>22||  222 | ||
|} | |} | ||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| − | |Check | + | | |
| − | |style="text-align:right;"|ולבחון אם החשבון אמת | + | ==== <span style=color:green>Check</span> ==== |
| + | |||
| + | | | ||
| + | |- | ||
| + | |To check if the calculation is correct: | ||
| + | |style="text-align:right;"|<big>ולבחון אם החשבון אמת</big> | ||
|- | |- | ||
| | | | ||
| Line 833: | Line 1,036: | ||
|- | |- | ||
| | | | ||
| − | : | + | :We sum up the upper 222, whose digits are 2, 2, 2; the result is 6. We write it next to them. |
| − | |style="text-align:right;"|קבצנו רכ"ב העליונים שסימנם ב'ב'ב' ועלו ו' וכתבנום אצלם | + | |style="text-align:right;"|קבצנו רכ"ב העליונים שסימנם ב'ב'ב' ועלו ו' וכתבנום אצלם |
| − | |||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{4+9+2+8+4=27\longrightarrow27\equiv_90}}</math> | + | :We multiply 6 by 6; the result is 36. |
| − | |style="text-align:right;"|וכן צריך להשאר הציפרא מן הסכום מן היוצא מן החשבון שהם מ"ט אלפים ורפ"ד אשר דמיונם הם ד' ט' ב' ח' ד' ועולה כ"ז< | + | |style="text-align:right;"|רבינו ו' על ו' ועלו ל"ו |
| − | ואם תשליכם ט"ט ישאר ציפרא נמצא שהחשבון הוא אמת | + | |- |
| + | | | ||
| + | :We cast out the nines; we are left with nothing. We write a zero between them. | ||
| + | |style="text-align:right;"|השלכנו אותם ט'ט' ולא נשאר בידינו ונתננו ציפרא באמצעם | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | :<math>\scriptstyle{\color{blue}{\begin{cases}\scriptstyle2+2+2=6\\\scriptstyle2+2+2=6\end{cases}\longrightarrow 6\times6=36\longrightarrow36\equiv_90}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :So, a zero should also remain from the sum of the calculation result, which is 49284, whose digits are 4, 9, 2, 8, 4. The sum is 27. | ||
| + | :<math>\scriptstyle{\color{blue}{4+9+2+8+4=27\longrightarrow27\equiv_90}}</math> | ||
| + | |style="text-align:right;"|וכן צריך להשאר הציפרא מן הסכום מן היוצא מן החשבון שהם מ"ט אלפים ורפ"ד אשר דמיונם הם ד' ט' ב' ח' ד' ועולה כ"ז‫<ref>כ"ז: MS Ithaca י"ח</ref> | ||
| + | |- | ||
| + | | | ||
| + | :If you cast out the nines, a zero remains. | ||
| + | |style="text-align:right;"|ואם תשליכם ט"ט ישאר ציפרא | ||
| + | |- | ||
| + | | | ||
| + | :We find that the calculation is correct. | ||
| + | |style="text-align:right;"|נמצא שהחשבון הוא אמת | ||
|- | |- | ||
| | | | ||
| − | === Units and tens by units, tens, and hundreds === | + | === <span style=color:green>Units and tens by units, tens, and hundreds</span> === |
| − | + | | | |
| − | |style="text-align:right;"|ואם רצינו לרבות ב' אותיות כנגד ג‫' | + | |- |
| + | |If we want to multiply two digits by three [digits]. | ||
| + | |style="text-align:right;"|<big>ואם רצינו</big> לרבות ב' אותיות כנגד ג‫' | ||
| + | |- | ||
| + | | | ||
| + | *For example, when we wish to [find the product of] 222 times 22, as you see in the diagram: | ||
| + | :<math>\scriptstyle22\times222</math> | ||
| + | |style="text-align:right;"|כגון שרצינו לומר <s>כ</s> ר'כ'ב' פעמים כ"ב כאשר תראה בצורה‫<ref>בצורה: MS Ithaca אותה</ref> | ||
|- | |- | ||
| | | | ||
| − | + | | | |
| − | | | ||
{|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
|- | |- | ||
| Line 865: | Line 1,092: | ||
|} | |} | ||
|- | |- | ||
| − | | | + | | |
| + | :We match them by [adding] a zero, so they are three digits by three digits and then the procedure is easier. | ||
|style="text-align:right;"|נשוה אותה עם הציפרא כדי שיהיו ג' אותיות כנגד ג' אותיות ויקל עשייתו | |style="text-align:right;"|נשוה אותה עם הציפרא כדי שיהיו ג' אותיות כנגד ג' אותיות ויקל עשייתו | ||
|- | |- | ||
| | | | ||
| − | === Units by units, tens, and hundreds === | + | === <span style=color:green>Units by units, tens, and hundreds</span> === |
| − | + | | | |
| − | |style="text-align:right;"|וכן אם רצינו לרבות אות אחת כנגד שלשה | + | |- |
| + | |If we want to multiply one digit by three [digits]. | ||
| + | |style="text-align:right;"|וכן אם רצינו לרבות אות‫<ref>אות: MS Ithaca אותו</ref> אחת כנגד שלשה | ||
|- | |- | ||
| − | | | + | |We match them also by [adding] zeros. |
|style="text-align:right;"|נשוה אותה עם הציפרא ג"כ | |style="text-align:right;"|נשוה אותה עם הציפרא ג"כ | ||
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle9\times222</math> | + | *As when we wish to know [the product of] 9 times 222. |
| − | |style="text-align:right;"|כגון שרצינו לדעת ט' פעמים ר'כ'ב' נכתוב אותם בזה הדרך כאשר תראה בצורה | + | :<math>\scriptstyle9\times222</math> |
| + | |style="text-align:right;"|כגון שרצינו לדעת ט' פעמים ר'כ'ב' <s>כ</s> | ||
| + | |- | ||
| + | | | ||
| + | :We write them this way, as you see in the diagram: | ||
| + | |style="text-align:right;"|נכתוב אותם בזה הדרך כאשר תראה בצורה | ||
| + | |- | ||
| + | | | ||
| + | | | ||
{|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
|- | |- | ||
| Line 895: | Line 1,133: | ||
|- | |- | ||
| | | | ||
| − | |||
| − | |style="text-align:right;"|אם נרצה לרבות ד' אותיות כנגד ד' אותיות | + | === <span style=color:green>Units, tens, hundreds, and thousands by units, tens, hundreds, and thousands</span> === |
| + | | | ||
| + | |- | ||
| + | |If we want to multiply four digits by four digits. | ||
| + | |style="text-align:right;"|<big>אם‫<ref>אם: MS Ithaca om.</ref> נרצה לרבות</big> ד'‫<ref>ד': MS Ithaca ג'</ref> אותיות כנגד ד'‫<ref>ד': MS Ithaca ג'</ref> אותיות | ||
| + | |- | ||
| + | | | ||
| + | *As when we wish to multiply 2222 times 2222. | ||
| + | :<math>\scriptstyle2222\times2222</math> | ||
| + | |style="text-align:right;"|כג כגון שרצינו לרבות ב' אלפים ור'כ'ב' פעמי' ב' אלפים ור'כ'ב‫' | ||
|- | |- | ||
| | | | ||
| − | + | :We multiply them seven times and write them seven times, in order that it will be clear to the quick and slow observer. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|נרבה אותם ז' פעמים ונכתוב אותם ז' פעמי'‫<ref>ונכתוב אותם ז' פעמי': MS Ithaca om.</ref> כדי שיתבאר למעיין הקודם והמתאחר‫<ref>Here ends this chapter in MS Ithaca</ref> |
|- | |- | ||
| − | | | + | | colspan="2"| |
| − | |||
| − | |||
{|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
|- | |- | ||
| Line 921: | Line 1,165: | ||
|- | |- | ||
| | | | ||
| + | :If we wish to multiply 2222 by 2222. | ||
|style="text-align:right;"|ואם רצינו לרבות ב' אלפי' ור'כ'ב' עם ב' אלפי' ור'כ'ב‫' | |style="text-align:right;"|ואם רצינו לרבות ב' אלפי' ור'כ'ב' עם ב' אלפי' ור'כ'ב‫' | ||
| + | |- | ||
| + | | colspan="2"| | ||
{|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
|- | |- | ||
| Line 937: | Line 1,184: | ||
|- | |- | ||
| | | | ||
| + | :We do as we did in the previous calculations. | ||
|style="text-align:right;"|נעשה כאשר עשינו בחשבונו' הקודמי‫' | |style="text-align:right;"|נעשה כאשר עשינו בחשבונו' הקודמי‫' | ||
|- | |- | ||
| | | | ||
| + | :The result of the calculation is 4937284. | ||
|style="text-align:right;"|ויעלה החשבון ד' אלפי אלפים ות'ת'ק'ל'ז' אלפי' ור'פ'ד‫' | |style="text-align:right;"|ויעלה החשבון ד' אלפי אלפים ות'ת'ק'ל'ז' אלפי' ור'פ'ד‫' | ||
|- | |- | ||
| | | | ||
| − | === Units, tens, hundreds, and thousands by units, tens, and hundreds === | + | === <span style=color:green>Units, tens, hundreds, and thousands by units, tens, and hundreds</span> === |
| − | + | | | |
| + | |- | ||
| + | |If we want to multiply four digits by three digits. | ||
|style="text-align:right;"|ואם רצינו לרבות ד' אותיות כנגד ג' אותיות | |style="text-align:right;"|ואם רצינו לרבות ד' אותיות כנגד ג' אותיות | ||
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle2222\times222</math> | + | *As when we want to multiply 2222 by 222. |
| + | :<math>\scriptstyle2222\times222</math> | ||
|style="text-align:right;"|כגון שרצינו לרבות ב' אלפים ור'כ'ב' כנגד ר'כ'ב‫' | |style="text-align:right;"|כגון שרצינו לרבות ב' אלפים ור'כ'ב' כנגד ר'כ'ב‫' | ||
|- | |- | ||
| − | | | + | | |
| + | :We match them by [adding] a zero, as you see in the diagram: | ||
|style="text-align:right;"|נשוה אותם עם הציפרא כאשר תראה בצורה | |style="text-align:right;"|נשוה אותם עם הציפרא כאשר תראה בצורה | ||
| + | |- | ||
| + | | | ||
| + | | | ||
{|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
|- | |- | ||
| Line 969: | Line 1,225: | ||
|- | |- | ||
| | | | ||
| + | :The result is 493284. | ||
|style="text-align:right;"|ועולים ת'צ'ג' אלפים ור'פ'ד‫' | |style="text-align:right;"|ועולים ת'צ'ג' אלפים ור'פ'ד‫' | ||
|- | |- | ||
| | | | ||
| − | === Units, tens, hundreds, and thousands by units and tens === | + | === <span style=color:green>Units, tens, hundreds, and thousands by units and tens</span> === |
| − | + | | | |
| + | |- | ||
| + | |If we want to multiply two digits by four [digits]. | ||
|style="text-align:right;"|וכן אם רצינו לרבות ב' אותיות כנגד ד‫' | |style="text-align:right;"|וכן אם רצינו לרבות ב' אותיות כנגד ד‫' | ||
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle2222\times22</math> | + | *As when we wish to multiply 2222 by 22. |
| + | :<math>\scriptstyle2222\times22</math> | ||
|style="text-align:right;"|כגון שרצינו לרבות ב' אלפים ור'כ'ב' כנגד כ"ב | |style="text-align:right;"|כגון שרצינו לרבות ב' אלפים ור'כ'ב' כנגד כ"ב | ||
|- | |- | ||
| − | | | + | | |
| + | :We match them by [adding] zeros, as you see in the diagram: | ||
|style="text-align:right;"|נשוה אותם עם הציפרא כאשר תראה בצורה | |style="text-align:right;"|נשוה אותם עם הציפרא כאשר תראה בצורה | ||
| + | |- | ||
| + | | | ||
| + | | | ||
{|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
|- | |- | ||
| Line 998: | Line 1,262: | ||
|- | |- | ||
| | | | ||
| + | :The result is 48884. | ||
|style="text-align:right;"|ועולים מ"ח אלפים ות'ת'פ'ד‫' | |style="text-align:right;"|ועולים מ"ח אלפים ות'ת'פ'ד‫' | ||
|- | |- | ||
| | | | ||
| − | === Units, tens, hundreds, and thousands by units === | + | === <span style=color:green>Units, tens, hundreds, and thousands by units</span> === |
| − | + | | | |
| + | |- | ||
| + | |If we want to multiply one digit by four digits. | ||
|style="text-align:right;"|וכן אם רצינו לרבות אות אחד כנגד כנגד ד' אותיות | |style="text-align:right;"|וכן אם רצינו לרבות אות אחד כנגד כנגד ד' אותיות | ||
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle2\times2222</math> | + | *As 2 by 2222. |
| + | :<math>\scriptstyle2\times2222</math> | ||
|style="text-align:right;"|כגון ב' עם ב' אלפים ור'כ'ב‫' | |style="text-align:right;"|כגון ב' עם ב' אלפים ור'כ'ב‫' | ||
|- | |- | ||
| − | | | + | | |
| + | :We match them by [adding] zeros, as you see in the diagram: | ||
|style="text-align:right;"|נשוה אותם עם הציפרא כאשר תראה בצורה | |style="text-align:right;"|נשוה אותם עם הציפרא כאשר תראה בצורה | ||
| + | |- | ||
| + | | | ||
| + | | | ||
{|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
|- | |- | ||
| Line 1,027: | Line 1,299: | ||
|- | |- | ||
| | | | ||
| + | :The result is 4444. | ||
|style="text-align:right;"|ועולים ד' אלפים ות'מ'ד‫' | |style="text-align:right;"|ועולים ד' אלפים ות'מ'ד‫' | ||
|- | |- | ||
| | | | ||
| − | === Units, tens, hundreds, thousands, and tens of thousands by units, tens, hundreds, thousands, and tens of thousands === | + | === <span style=color:green>Units, tens, hundreds, thousands, and tens of thousands by units, tens, hundreds, thousands, and tens of thousands</span> === |
| − | + | | | |
| + | |- | ||
| + | |If we want to multiply five digits by five digits. | ||
|style="text-align:right;"|אם נרצה לרבות ה' אותיות כנגד ה' אותיות | |style="text-align:right;"|אם נרצה לרבות ה' אותיות כנגד ה' אותיות | ||
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle22222\times22222</math> | + | *As when we wish to know [the product of] 22222 by 22222. |
| + | :<math>\scriptstyle22222\times22222</math> | ||
|style="text-align:right;"|כגון שרצינו לדעת כ"ב אלפים ור'כ'ב' כ"ב אלפי' ור'כ'ב‫' | |style="text-align:right;"|כגון שרצינו לדעת כ"ב אלפים ור'כ'ב' כ"ב אלפי' ור'כ'ב‫' | ||
|- | |- | ||
| − | | | + | | |
| − | + | :We multiply them nine times and this is the method of multiplication: | |
| − | |style="text-align:right;"|נרבה אותם ט' פעמים וזה דרך רבויים | + | |style="text-align:right;"|נרבה אותם ט' פעמים וזה דרך רבויים |
| − | ונכתוב אותם ט' פעמי' כדי שיתבאר למעיין הקודם הקודם והמתאחר | + | |- |
| + | | | ||
| + | :We write them nine times, in order that it will be clear to the quick and slow observer. | ||
| + | |style="text-align:right;"|ונכתוב אותם ט' פעמי' כדי שיתבאר למעיין הקודם הקודם והמתאחר | ||
| + | |- | ||
| + | | colspan="2"| | ||
{|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
|- | |- | ||
| Line 1,059: | Line 1,340: | ||
| | | | ||
| − | === Units, tens, hundreds, thousands, tens of thousands, and hundreds of thousands by units, tens, hundreds, thousands, tens of thousands, and hundreds of thousands === | + | === <span style=color:green>Units, tens, hundreds, thousands, tens of thousands, and hundreds of thousands by units, tens, hundreds, thousands, tens of thousands, and hundreds of thousands</span> === |
| − | + | | | |
| + | |- | ||
| + | |If we want to multiply six digits by six digits. | ||
|style="text-align:right;"|ואם נרצה לרבות ו' אותיות כנגד ו' אותיות | |style="text-align:right;"|ואם נרצה לרבות ו' אותיות כנגד ו' אותיות | ||
|- | |- | ||
| − | | | + | |We multiply them 11 times. |
|style="text-align:right;"|נרבה אותם י"א פעמי‫' | |style="text-align:right;"|נרבה אותם י"א פעמי‫' | ||
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle222222\times222222</math> | + | *As when we wish to multiply 222222 by 222222. |
| + | :<math>\scriptstyle222222\times222222</math> | ||
|style="text-align:right;"|כגון שרצינו לרבות ר'כ'ב' אלפי' ור'כ'ב' ר'כ'ב' אלפים ור'כ'ב‫' | |style="text-align:right;"|כגון שרצינו לרבות ר'כ'ב' אלפי' ור'כ'ב' ר'כ'ב' אלפים ור'כ'ב‫' | ||
|- | |- | ||
| | | | ||
| + | :We multiply them 11 times, in order that it will be clear to the quick and slow observer. | ||
|style="text-align:right;"|ונרבה אותם י"א פעמי' כדי שיתבאר למעיין הקודם והמתאחר | |style="text-align:right;"|ונרבה אותם י"א פעמי' כדי שיתבאר למעיין הקודם והמתאחר | ||
| − | |||
|} | |} | ||
| + | |||
{|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
|- | |- | ||
| Line 1,087: | Line 1,372: | ||
|- | |- | ||
|} | |} | ||
| − | |||
{| | {| | ||
|- | |- | ||
| | | | ||
| − | == | + | == The Method of Summing Up Separate Numbers Called Congregare or Riunire == |
| − | + | |style="width:45%;text-align:right;"|<big>דרך קיבוץ חשבונות נפרדים הנקראים קונגרייג</big> או ריונרייי | |
|- | |- | ||
| − | | | + | |If we want to sum up separate numbers and bring them into one sum, we do this way: we write them successively one beneath the other: the units beneath the units, the tens corresponding the tens, the hundreds corresponding the hundreds and the thousands corresponding the thousands. |
| − | |style="text-align:right;"|אם רצינו לקבץ חשבונות נפרדים ולהביאם לסכום אחד | + | |style="text-align:right;"|אם רצינו לקבץ חשבונות נפרדים ולהביאם לסכום אחד נעשה על זאת הדרך שנכתוב אותם על הסדר זה תחת זה האחדי' תחת האחדים והעשרות נכח העשרות והמאות נכח המאות והאלפים נכח האלפים |
|- | |- | ||
| − | | | + | |We start summing the units and write [their sum]. |
| − | |||
| − | |||
| − | |||
| − | |||
|style="text-align:right;"|ונתחיל לקבץ האחדי' ולכותבם | |style="text-align:right;"|ונתחיל לקבץ האחדי' ולכותבם | ||
|- | |- | ||
| − | | | + | |If [the sum] is more than ten, we write the units and keep the tens. |
| − | |||
|style="text-align:right;"|אם יעלו על עשר נכתוב האחדים ונחזיק בידינו העשרות | |style="text-align:right;"|אם יעלו על עשר נכתוב האחדים ונחזיק בידינו העשרות | ||
|- | |- | ||
| − | | | + | |Then, we sum the hundreds, then the thousands, as you see in this diagram: |
|style="text-align:right;"|אחרי כן נקבץ המאות אחרי כן נקבץ האלפים כמו שתראה בצורה הזאת | |style="text-align:right;"|אחרי כן נקבץ המאות אחרי כן נקבץ האלפים כמו שתראה בצורה הזאת | ||
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle12345+67891+23456+78912+34567</math> | + | *Example: we wish to sum up 12345 with 67891, 23456, 78912, and 34567. |
| − | |style="text-align:right;"|דמיון זה רצינו לקבץ י"ב אלפים וש'מ'ה' וס"ז אלפי' ות'ת'צ'א' וכ"ג אלפים ת'נ'ו' וע"ח אלפים ות'ת'ק'י'ב' ול"ד אלפים ות'ק'ס'ז' | + | :<math>\scriptstyle12345+67891+23456+78912+34567</math> |
| + | |style="text-align:right;"|‫<ref>33r</ref>דמיון זה רצינו לקבץ י"ב‫<ref>י"ב: MS Ithaca י"ג</ref> אלפים וש'מ'ה' וס"ז אלפי' ות'ת'צ'א' וכ"ג אלפים ת'נ'ו' וע"ח אלפים ות'ת'ק'י'ב' ול"ד אלפים ות'ק'ס'ז'‫<ref>תקס"ז: MS Ithaca תתקס"ז</ref> | ||
|- | |- | ||
| | | | ||
| Line 1,160: | Line 1,440: | ||
|- | |- | ||
| | | | ||
| − | :: | + | :We start summing the units and say: 5, 1, 6, 2, 7 are 21. We write 1 and keep 2. |
| − | |style="text-align:right;"|נתחיל לקבץ האחדים ונאמר ה' וא' וו' וב' וז' הם כ"א נכתוב א' ונחזי' בידינו ב' | + | :<math>\scriptstyle{\color{blue}{5+1+6+2+7={\color{green}{2}}1}}</math> |
| + | |style="text-align:right;"|נתחיל לקבץ האחדים ונאמר ה' וא' וו' וב' וז' הם כ"א נכתוב א' ונחזי' בידינו ב‫' | ||
|- | |- | ||
| | | | ||
| − | :: | + | :Then, we sum the tens and say: 4, 9, 5, 1, 6 are 25; with the 2 we have kept, they are 27. We write 7 and keep 2. |
| − | |style="text-align:right;"|נקבץ אחרי כן העשרות ונאמר ד' וט' וה' וא' וו' הם כ"ה וב' | + | :<math>\scriptstyle{\color{blue}{\left(4+9+5+1+6\right)+{\color{green}{2}}=25+2={\color{green}{2}}7}}</math> |
| + | |style="text-align:right;"|נקבץ אחרי כן העשרות ונאמר ד' וט' וה' וא' וו' הם כ"ה וב' שה<sup>ח</sup>זקנו בידינו הם כ"ז נכתו' ז' ונחזיק בידינו ב‫' | ||
|- | |- | ||
| | | | ||
| − | :: | + | :Then, we sum the hundreds and say: 3, 8, 4, 9, 5 are 29; with the 2 we have kept, they are 31. We write 1 and keep 3. |
| − | |style="text-align:right;"|נקבץ אחרי כן המאות ונאמ' ג' וח' וד' וט' וה' הם כ"ט וב' שהחזקנו בידינו הרי ל"א נכתוב א' ונחזיק בידינו ג' | + | :<math>\scriptstyle{\color{blue}{\left(3+8+4+9+5\right)+{\color{green}{2}}=29+2={\color{green}{3}}1}}</math> |
| + | |style="text-align:right;"|נקבץ אחרי כן המאות ונאמ' ג' וח' וד' וט' וה' הם כ"ט וב' שהחזקנו בידינו הרי ל"א נכתוב א' ונחזיק בידינו ג‫' | ||
|- | |- | ||
| | | | ||
| − | :: | + | :Then, we sum the thousands and say: 2, 7, 3, 8, 4 are 24; with the 3 we have kept, they are 27. We write 7 and keep 2. |
| − | |style="text-align:right;"|נקבץ אחרי כן האלפים ונאמר ב' וז' וג' וח' וד' הם כ"ד וג' שהחזקנו בידינו הרי כ"ז ונכתוב ז' ונחזיק בידינו ב' | + | :<math>\scriptstyle{\color{blue}{\left(2+7+3+8+4\right)+{\color{green}{3}}=24+3={\color{green}{2}}7}}</math> |
| + | |style="text-align:right;"|נקבץ אחרי כן האלפים ונאמר ב' וז' וג' וח' וד' הם כ"ד וג' שהחזקנו בידינו הרי כ"ז ונכתוב ז' ונחזיק בידינו ב‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | :Then, we sum the tens of thousands and say: 1, 6, 2, 7, 3 are 19; with the 2 we have kept, they are 21. We write everything, because we do not have another number to sum. |
| − | |style="text-align:right;"|נקבץ אחר זה העשרות מן האלפים | + | :<math>\scriptstyle{\color{blue}{\left(1+6+2+7+3\right)+{\color{green}{2}}=19+2=21}}</math> |
| + | |style="text-align:right;"|נקבץ אחר זה העשרות מן האלפים ונאמ' א' וו' וב'‫<ref>וב': MS Ithaca om.</ref> וז' וג' הם י"ט‫<ref>הם י"ט: MS Ithaca om.</ref> וב' שהחזקנו בידינו הרי כ"א נכתוב אותם כלם כי אין לנו לקבץ חשבון אחר | ||
|- | |- | ||
| | | | ||
| + | :The total result is 217171. | ||
|style="text-align:right;"|עלו כלם רי"ז אלפים וקע"א | |style="text-align:right;"|עלו כלם רי"ז אלפים וקע"א | ||
|- | |- | ||
| − | | | + | |If we want to sum up numbers, some of which have three digits, some have four, some have two, and some have more or less: |
| − | + | |style="text-align:right;"|<big>ואם רצינו</big> לקבץ חשבונות נפרדים שקצתם הם מג' אותיות וקצתם מד' וקצתם מב' וקצתם מיותר או פחות | |
| − | |style="text-align:right;"|ואם רצינו לקבץ חשבונות נפרדים שקצתם הם מג' אותיות וקצתם מד' וקצתם מב' וקצתם מיותר או פחות | ||
|- | |- | ||
| − | | | + | |We always write the units corresponding to the units, the tens corresponding to the tens and so on and we match them by [adding] zeros to make the procedure easier. |
| − | |||
|style="text-align:right;"|נכתוב לעולם האחדים נוכח האחדי' והעשרות נוכח העשרות וכן כלם ונשוה אותם עם הציפרי כדי שיקל העניין | |style="text-align:right;"|נכתוב לעולם האחדים נוכח האחדי' והעשרות נוכח העשרות וכן כלם ונשוה אותם עם הציפרי כדי שיקל העניין | ||
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle12345+06789+00123+45600+80912+03406</math> | + | *Example: we wish to sum up 12345 with 6789, 123, 45600, 80912, and 3406. |
| − | |style="text-align:right;"|דמיון זה רצינו לקבץ י"ב אלפים וש'מ'ה' וו' אלפים ות"שפ"ט וקכ"ג ומ"ה אלפים ות"ר ופ' אלפי' ות"תקי"ב וג' אלפים ות"ו | + | :<math>\scriptstyle12345+06789+00123+45600+80912+03406</math> |
| + | |style="text-align:right;"|<big>דמיון זה</big> רצינו לקבץ י"ב אלפים וש'מ'ה' וו' אלפים ות"שפ"ט וקכ"ג ומ"ה אלפים ות"ר ופ' אלפי' ות"תקי"ב וג' אלפים ות"ו | ||
|- | |- | ||
| | | | ||
| Line 1,238: | Line 1,523: | ||
|- | |- | ||
| | | | ||
| − | :: | + | :We start summing the units and say: 5, 9, 3, 2, 6 are 25. We write [5] and keep 2. |
| − | |style="text-align:right;"|התחלנו לקבץ האחדים ואמרנו ה' וט' וג' וב' וו' הם כ"ה כתבנו כ"ה והחזקנו בידינו ב' | + | :<math>\scriptstyle{\color{blue}{5+9+3+2+6={\color{green}{2}}5}}</math> |
| + | |style="text-align:right;"|התחלנו לקבץ האחדים ואמרנו ה' וט' וג' וב' וו' הם כ"ה כתבנו כ"ה והחזקנו בידינו ב‫' | ||
|- | |- | ||
| | | | ||
| − | :: | + | :Then, we sum the tens and say: 4, 8, 2, 1 are 15; with the 2 we have kept, they are 17. We write 7 and keep 1. |
| − | |style="text-align:right;"|קבצנו אחר כן העשרות ואמרנו ד' וח' וב' וא' הם ט"ו ועם הב' שהחזקנו בידינו הם י"ז כתבנו ז' והחזקנו בידינו א' | + | :<math>\scriptstyle{\color{blue}{\left(4+8+2+1\right)+{\color{green}{2}}=15+2={\color{green}{1}}7}}</math> |
| + | |style="text-align:right;"|קבצנו אחר כן‫<ref>אחר כן: MS Ithaca בידינו</ref> העשרות ואמרנו ד' וח' וב' וא' הם ט"ו ועם הב' שהחזקנו בידינו הם י"ז כתבנו ז' והחזקנו בידינו א‫' | ||
|- | |- | ||
| | | | ||
| − | :: | + | :Then, we sum the hundreds and say: 3, 7, 1, 6, 9, 4 are 30; with the 1 we have kept, they are 31. We write 1 and keep 3. |
| − | |style="text-align:right;"|קבצנו אחרי כן המאות ואמרנו ג' וז' וא' וו' וט' וד' הם ל' ועם הא' שהחזקנו בידינו הם ל"א כתבנו א' והחזקנו בידינו ג' | + | :<math>\scriptstyle{\color{blue}{\left(3+7+1+6+9+4\right)+{\color{green}{1}}=30+1={\color{green}{3}}1}}</math> |
| + | |style="text-align:right;"|קבצנו אחרי כן המאות ואמרנו ג' וז' וא' וו' וט' וד' הם ל' ועם הא' שהחזקנו בידינו הם ל"א כתבנו א' והחזקנו בידינו ג‫' | ||
|- | |- | ||
| | | | ||
| − | :: | + | :Then, we sum the thousands and say: 2, 6, 5, 3 are 16; with the 3 we have kept, they are 19. We write 9 and keep 1. |
| − | |style="text-align:right;"|קבצנו אחרי כן האלפים ואמרנו ב' וו' וה' וג' הם י"ו ועם הג' שהחזקנו בידינו הם י"ט כתבנו ט' והחזקנו בידינו א' | + | :<math>\scriptstyle{\color{blue}{\left(2+6+5+3\right)+{\color{green}{3}}=16+3={\color{green}{1}}9}}</math> |
| + | |style="text-align:right;"|קבצנו אחרי כן האלפים ואמרנו ב' וו' וה' וג' הם י"ו ועם הג' שהחזקנו בידינו הם י"ט כתבנו ט' והחזקנו בידינו א‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | :Then, we sum the tens of thousands and say: 1, 4, 8 are 13; with the 1 we have kept, they are 14. We write 14, because we have summed everything. |
| − | |style="text-align:right;"|קבצנו אחר זה העשרות מן האלפי' ואמרנו א' וד' וח' הם י"ג ועם א' שהחזקנו בידינו הם י"ד וכתבנו י"ד כי מעתה קבצנו הכל | + | :<math>\scriptstyle{\color{blue}{\left(1+4+8\right)+{\color{green}{1}}=13+1=14}}</math> |
| + | |style="text-align:right;"|קבצנו אחר זה העשרות מן האלפי' ואמרנו א' וד' וח' הם י"ג ועם א' שהחזקנו בידינו הם י"ד‫<ref>ועם... י"ד: MS Ithaca om.</ref> וכתבנו י"ד כי מעתה קבצנו הכל | ||
|- | |- | ||
| | | | ||
| + | :Their sum is 149175. | ||
|style="text-align:right;"|ועולה סכומם קמ"ט אלפים וקע"ה | |style="text-align:right;"|ועולה סכומם קמ"ט אלפים וקע"ה | ||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | == | + | == The Method of Subtracting a Number from a Number Called Sottrarre == |
| − | + | |style="text-align:right;"|<big>דרך הוצאת מספר ממספר הנק' סורטראיירי</big> | |
|- | |- | ||
| − | | | + | |If we want to subtract a number from a number: |
| − | + | |style="text-align:right;"|אם רצינו לגרוע מספר ממספר | |
| − | |style="text-align:right;"| | + | |- |
| + | |If all the [digits] of the larger number, from which you want to subtract another number, are greater than the [digits] of that number, the procedure is easy. | ||
| + | |style="text-align:right;"|אם המספר הגדול אשר אתה רוצה לגרוע ממנו מספר אחר כל חלקיו גדולי' מחלקי המספר הוא דרך נקל | ||
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle987654-645232</math> | + | *Example: we wish to subtract 645232 from 987654. |
| − | |style="text-align:right;"|דמיון זה רצינו לגרוע מתת"קפ"ז אלפים ותרנ"ד ת"רמ"ה אלפים ורל"ב | + | :<math>\scriptstyle987654-645232</math> |
| + | |style="text-align:right;"|<big>דמיון זה</big> רצינו לגרוע מתת"קפ"ז אלפים ותרנ"ד ת"רמ"ה אלפים ורל"ב | ||
|- | |- | ||
| | | | ||
| Line 1,295: | Line 1,586: | ||
|} | |} | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"|נעשה על זה הדרך | + | :We do this way: |
| + | |style="text-align:right;"|נעשה על זה הדרך | ||
|- | |- | ||
| | | | ||
| − | :: | + | :We start from the units and say: subtract 2 from 4; 2 remains. We write 2 beneath them. |
| − | |style="text-align:right;"|ונאמר מד' הוצא ב' ישארו ב' ונכתוב ב' תחתיהם | + | :<math>\scriptstyle{\color{blue}{4-2=2}}</math> |
| + | |style="text-align:right;"|נתחיל מן האחדים ונאמר מד' הוצא ב' ישארו ב' ונכתוב ב' תחתיהם | ||
|- | |- | ||
| | | | ||
| − | : | + | :Subtract 3 from 5; 2 remains. Write 2 beneath them. |
| + | :<math>\scriptstyle{\color{blue}{5-3=2}}</math> | ||
|style="text-align:right;"|ומן ה' הוצא ג' ישארו ב' ותכתוב ב' תחתיהם | |style="text-align:right;"|ומן ה' הוצא ג' ישארו ב' ותכתוב ב' תחתיהם | ||
|- | |- | ||
| | | | ||
| − | : | + | :Subtract 2 from 6; 4 remains. Write 4 beneath them. |
| + | :<math>\scriptstyle{\color{blue}{6-2=4}}</math> | ||
|style="text-align:right;"|ומן ו' הוצא ב' ישארו ד' ותכתוב ד' תחתיהם | |style="text-align:right;"|ומן ו' הוצא ב' ישארו ד' ותכתוב ד' תחתיהם | ||
|- | |- | ||
| | | | ||
| − | : | + | :Subtract 5 from 7; 2 remains. Write 2 beneath them. |
| + | :<math>\scriptstyle{\color{blue}{7-5=2}}</math> | ||
|style="text-align:right;"|ומן ז' תוצא ה' ישארו ב' ונכתוב ב' תחתיהם | |style="text-align:right;"|ומן ז' תוצא ה' ישארו ב' ונכתוב ב' תחתיהם | ||
|- | |- | ||
| | | | ||
| − | : | + | :Subtract 4 from 8; 4 remains. Write 4 beneath them. |
| − | |style="text-align:right;"|ומן ח' הוצא ד' ישארו ד' ונכתוב ד' תחתיהם | + | :<math>\scriptstyle{\color{blue}{8-4=4}}</math> |
| + | |style="text-align:right;"|ומן ח' הוצא ד' ישארו ד' ונכתוב ד' תחתיהם‫<ref>ומן ז'... ד' תחתיהם: MS Ithaca om.</ref> | ||
|- | |- | ||
| | | | ||
| − | : | + | :Subtract 6 from 9; 3 remains. Write 3 beneath them. |
| + | :<math>\scriptstyle{\color{blue}{9-6=3}}</math> | ||
|style="text-align:right;"|ומן ט' הוצא ו' ישארו ג' ותכתוב ג' תחתיהם | |style="text-align:right;"|ומן ט' הוצא ו' ישארו ג' ותכתוב ג' תחתיהם | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|הרי שאם נוציא מת"תק'פ'ז' אלפים ות"רנ"ד ת"רמ"ה אלפים ורל"ב ישארו ש'מ'ב' אלפים ות'כ'ב' וזהו דרך נקל | + | :So, if we subtract 645232 from 987654, 342422 remains and this procedure is easy. |
| + | |style="text-align:right;"|הרי שאם נוציא מת"תק'פ'ז' אלפים <s>ת</s> ות"רנ"ד ת"רמ"ה אלפים <s>ול</s> ורל"ב ישארו ש'מ'ב' אלפים ות'כ'ב' וזהו דרך נקל | ||
|- | |- | ||
| − | |Check: | + | |<span style="color:green">'''Check - addition:'''</span> If you want to check if your calculation is correct, sum the digits you subtracted with the digits that remained and if [their sums] are the same as the digits from which you subtracted the other digits, it is correct. |
| − | |style="text-align:right;"|ואם תרצה לבחון אם החשבון שעשית הוא אמתי תקבץ המספרים שהוצאת עם המספרים שנשארו ואם יסכימו עם המספרים שהוצאת המספרים האחרי' ההם הוא אמתי | + | |style="text-align:right;"|<big>ואם תרצה לבחון</big> אם החשבון שעשית הוא אמתי תקבץ המספרים שהוצאת עם המספרים שנשארו ואם יסכימו עם המספרים שהוצאת המספרים האחרי' ההם הוא אמתי |
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle645232+342422=987654</math> | + | :Example: We sum 2 with 2; it is 4. We sum 3 with 2; it is 5. We sum 2 with 4; it is 6. We sum 5 with 2; it is 7. We sum 4 with 4; it is 8. We sum 6 with 3; it is 9. They are the same as the greater digits from which you subtracted the smaller digits. This indicates that the calculation is correct. |
| − | |style="text-align:right;"|דמיון זה קבצנו ב' עם ב' והיו ד' וקבצנו ג' וב' והיו ה' וקבצנו ב' עם ד' והיו ו' וקבצנו ה' עם ב' והיו ז' וקבצנו ד' עם ד' והיו ח' וקבצנו ו' עם ג' והיו ט' והם מסכימים עם המספרים הגדולים אשר הוצאת ממספר הקטנים מהם וזה יורה כי החשבון הוא אמתי | + | :<math>\scriptstyle645232+342422=987654</math> |
| + | |style="text-align:right;"|<big>דמיון</big> זה קבצנו ב' עם ב' והיו ד' וקבצנו ג' וב' והיו ה' וקבצנו ב' עם ד' והיו ו' וקבצנו ה' עם ב' והיו ז' וקבצנו ד' עם ד' והיו ח' וקבצנו ו' עם ג' והיו ט' והם מסכימים עם המספרים הגדולים אשר הוצאת ממספר הקטנים מהם וזה יורה כי החשבון הוא אמתי | ||
|- | |- | ||
| − | | | + | |If you want to subtract a number from a number, but some of the digits of the smaller number that you want to subtract from the greater number are greater than some of the [corresponding] digits of the greater number, you should use another procedure: |
| − | + | |style="text-align:right;"|<big>אמנם</big> אם תרצה לגרוע מספר ממספר ויהיו קצת חלקי המספר הקטן שאתה רוצה לפחות מהמספר הגדול גדולים מקצת מספר חלקי המספר הגדול אתה צריך לעשות על דרך אחרת | |
| − | |style="text-align:right;"|אמנם אם תרצה לגרוע מספר ממספר ויהיו קצת חלקי המספר הקטן שאתה רוצה לפחות מהמספר הגדול גדולים מקצת מספר חלקי המספר הגדול אתה צריך לעשות על דרך אחרת | ||
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle93432-27654</math> | + | *Example: we wish to subtract 27654 from 93432. |
| − | |style="text-align:right;"|דמיון זה רצינו לגרוע מצ"ג אלפים ותל"ב כ"ז אלפים ות"רנ"ד | + | :<math>\scriptstyle93432-27654</math> |
| + | |style="text-align:right;"|<big>דמיון זה</big> רצינו לגרוע מצ"ג אלפים ותל"ב כ"ז אלפים ות"רנ"ד | ||
|- | |- | ||
| | | | ||
| Line 1,359: | Line 1,659: | ||
|- | |- | ||
| | | | ||
| − | : | + | :We start from the units and say: subtract 4 from 2; we cannot. |
| + | |style="text-align:right;"|נתחיל מן האחדי' ותאמר מב' תוצי' ד' לא נוכל | ||
|- | |- | ||
| − | | | + | | |
| + | :We subtract 1 from the 3 next to it and add it to the 2; they are 12. | ||
| + | |style="text-align:right;"|נפחות א' מן הג' שבצדה ונחבר אותה אל הב' ויהיו י"ב | ||
|- | |- | ||
| − | |2765<span style="color:red">4</span>||276<span style="color:red">5</span>4||27<span style="color:red">6</span>54 | + | | |
| + | :We say: subtract 4 from 12; 8 remains. We write it. | ||
| + | |style="text-align:right;"|ונאמר מי"ב הוצא ד' ישארו ח'‫<ref>ישארו ח': MS Ithaca marg.</ref> ונכתבם | ||
| + | |- | ||
| + | | | ||
| + | :We return to the 3, from which we have subtracted 1; 2 remains. | ||
| + | |style="text-align:right;"|ונחזור אל הג' שפחתנו ממנו א' וישארו ב‫' | ||
| + | |- | ||
| + | | | ||
| + | :We say: subtract 5 from 2; we cannot. | ||
| + | |style="text-align:right;"|ונאמר מב' הוצא ה' לא נוכל | ||
| + | |- | ||
| + | | | ||
| + | :We subtract 1 from the 4 next to it and say: subtract 5 from 12; 7 remains. We write it. | ||
| + | |style="text-align:right;"|נפחות א' מהד' אשר בצדה ונאמר מי"ב הוצא ה' ישארו ז' ונכתבם | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | :{| | ||
| + | |- | ||
| + | |9343<span style="color:red">2</span>||rowspan="3"|<math>\scriptstyle\xrightarrow{\begin{align}&\scriptstyle{\color{red}{3-1}}={\color{green}{2}}\\&\scriptstyle{\color{green}{1}}{\color{red}{2-4}}={\color{blue}{8}}\\\end{align}}</math>||934<span style="color:red">3</span>2||rowspan="3"|<math>\scriptstyle\xrightarrow{\begin{align}&\scriptstyle{\color{red}{4-1}}={\color{green}{3}}\\&\scriptstyle{\color{green}{12}}{\color{red}{-5}}={\color{blue}{7}}\\\end{align}}</math>||93<span style="color:red">4</span>32 | ||
| + | |- | ||
| + | |2765<span style="color:red">4</span>||276<span style="color:red">5</span>4||27<span style="color:red">6</span>54 | ||
|- | |- | ||
| ||    <span style="color:#0000FF>8</span>||   <span style="color:#0000FF>7</span>8 | | ||    <span style="color:#0000FF>8</span>||   <span style="color:#0000FF>7</span>8 | ||
|} | |} | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| + | :We return to the 4, from which we have subtracted 1; 3 remains. | ||
| + | |style="text-align:right;"|ונחזור אל הד' שפחתנו ממנה א' ונשארו ג‫' | ||
| + | |- | ||
| + | | | ||
| + | :We say: subtract 6 from 3; we cannot. | ||
| + | |style="text-align:right;"|ונאמר מג' הוצא ו' ולא נוכל | ||
| + | |- | ||
| + | | | ||
| + | :We subtract 1 from the 3 next to it and say: subtract 6 from 13; 7 remains. We write it. | ||
| + | |style="text-align:right;"|נפחות א' מן הג' אשר בצדה ונאמר מי"ג הוצא ו' וישארו ז' ונכתבם | ||
| + | |- | ||
| + | | | ||
| + | :We return to the 3, from which we have subtracted 1; 2 remains. | ||
| + | |style="text-align:right;"|ונחזור אל הג' שפחתנו ממנו א' ונשארו ב‫' | ||
| + | |- | ||
| + | | | ||
| + | :We say: subtract 7 from 2; we cannot. | ||
| + | |style="text-align:right;"|ונאמר מב' הוצא ז' לא נוכל | ||
| + | |- | ||
| + | | | ||
| + | :We subtract 1 from the 9 next to it and say: subtract 7 from 12; 5 remains. We write it. | ||
| + | |style="text-align:right;"|נפחות א' מן הט' אשר בצדה ונאמר מי"ב הוצא ז' ישארו ה' ונכתבם | ||
| + | |- | ||
| + | | | ||
| + | :We return to the 9, from which we have subtracted 1; 8 remains. | ||
| + | |style="text-align:right;"|ונחזור אל הט' אשר פחתנו ממנה א' ונשארו ח‫' | ||
| + | |- | ||
| + | | | ||
| + | :We say: subtract 2 from 8; 6 remains. We write it. | ||
| + | |style="text-align:right;"|ונאמר מח' הוצא ב' ישארו ו' ונכתבנו | ||
| + | |- | ||
| + | | | ||
| + | :Hence, we subtract 27654 from 93432 and 65778 remains | ||
| + | |style="text-align:right;"|הרי שהוצאנו מצ"ג אלפים ותל"ב כ"ז אלפים ות"רנ"ד‫<ref>ותרנ"ד: MS Ithaca ותרנ"ב</ref> ונשארו ס"ה אלפים ות"שע"ח | ||
| + | |- | ||
| + | | colspan="2"| | ||
:::{| | :::{| | ||
|- | |- | ||
| Line 1,379: | Line 1,737: | ||
|  <span style="color:#0000FF>7</span>78|| <span style="color:#0000FF>5</span>778||<span style="color:#0000FF>6</span>5778 | |  <span style="color:#0000FF>7</span>78|| <span style="color:#0000FF>5</span>778||<span style="color:#0000FF>6</span>5778 | ||
|} | |} | ||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| − | |Check: | + | |<span style="color:green">'''Check - addition:'''</span> If you sum what you subtracted from the mentioned number with the remainder, it will be the same as the original number. |
|style="text-align:right;"|והנה אם תקבץ מה שהוצאתו מהמספר הנזכר עם הנשאר יהיה כמספר הראשון | |style="text-align:right;"|והנה אם תקבץ מה שהוצאתו מהמספר הנזכר עם הנשאר יהיה כמספר הראשון | ||
|- | |- | ||
| | | | ||
| − | + | :<math>\scriptstyle27654+65778=93432</math> | |
| − | |style="text-align:right;"|וזה שאם נקבץ ד' וח' יהיו י"ב ונכתוב ב' ונחזיק בידינו א' | + | :If we sum 4 with 8; they are 12. We write 2 and keep 1. |
| − | ואם נקבץ ה' וז' יהיו י"ב ועם הא' שנשארה בידינו יהיו י"ג ונכתוב ג' ונחזיק א' | + | |style="text-align:right;"|וזה שאם נקבץ ד' וח' יהיו י"ב ונכתוב ב' ונחזיק בידינו א‫' |
| − | ואם נקבץ ו' וז' יהיו י"ג וא' שנשאר בידינו הרי י"ד ונכתוב ד' ונחזיק א' | + | |- |
| − | ואם נקבץ ז' וה' יהיו י"ב וא' שנשאר בידינו הרי י"ג ונכתוב ג' ונחזיק א' | + | | |
| − | + | :If we sum 5 with 7; they are 12. With the 1 we have left, they are 13. We write 3 and keep 1. | |
| − | + | |style="text-align:right;"|ואם נקבץ ה' וז' יהיו י"ב ועם הא' שנשארה בידינו יהיו י"ג ונכתוב ג' ונחזיק א‫' | |
| + | |- | ||
| + | | | ||
| + | :If we sum 6 with 7; they are 13. With the 1 we have left, they are 14. We write 4 and keep 1. | ||
| + | |style="text-align:right;"|ואם נקבץ ו' וז' יהיו י"ג וא' שנשאר בידינו הרי י"ד ונכתוב ד' ונחזיק א‫' | ||
| + | |- | ||
| + | | | ||
| + | :If we sum 7 with 5; they are 12. With the 1 we have left, they are 13. We write 3 and keep 1. | ||
| + | |style="text-align:right;"|ואם נקבץ ז' וה' יהיו י"ב וא' שנשאר בידינו הרי י"ג ונכתוב ג' ונחזיק א‫' | ||
|- | |- | ||
| − | | | + | | |
| − | + | :If we sum 2 with 6; they are 8. With the 1 we have left, they are 9. We write it. | |
| + | |style="text-align:right;"|ואם נקבץ ב' וו' יהיו ח' וא' שנשאר בידינו הרי ט' ונכתבם | ||
| + | |- | ||
| + | | | ||
| + | :So, the final number is the same as the original [number]. | ||
| + | |style="text-align:right;"|הרי שהמספר האחרון שוה עם הראשון | ||
| + | |- | ||
| + | |The method of division. | ||
| + | |style="text-align:right;"|דרך חילוק | ||
|- | |- | ||
| | | | ||
| − | == | + | == The Method of Dividing a Number by a Number Called Dividere or Partire == |
| − | + | |style="text-align:right;"|‫<ref>34r</ref><big>דרך חילוק מספר על מספר הנק' דיבי' או פארטיר‫'</big> | |
|- | |- | ||
| − | | | + | |If we want to divide a larger number by a smaller number: |
| − | |style="text-align:right;"|אם נרצה לחלוק מספר גדול על מספר קטן אם כל חלקי המספר הגדול הם גדולי מהמספר שאתה רוצה לחלק אותם בו הוא דרך נקל | + | |style="text-align:right;"|אם נרצה לחלוק מספר גדול על מספר קטן |
| + | |- | ||
| + | |If all the digits of the large number are larger than the number by which you want to divide them, the procedure is easy. | ||
| + | |style="text-align:right;"|אם כל חלקי המספר הגדול הם גדולי מהמספר שאתה רוצה לחלק אותם בו הוא דרך נקל | ||
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle98756\div4</math> | + | *Example: we wish to divide 98756 by 4. |
| − | |style="text-align:right;"|דמיון זה רצינו לחלק צ"ח אלפים ות'ש'נ'ו' על ד' | + | :<math>\scriptstyle98756\div4</math> |
| + | |style="text-align:right;"|<big>דמיון זה</big> רצינו לחלק צ"ח אלפים ות'ש'נ'ו' על ד‫' | ||
|- | |- | ||
| | | | ||
| Line 1,440: | Line 1,814: | ||
|- | |- | ||
| | | | ||
| − | : | + | :We do this way: |
| + | |style="text-align:right;"|נעשה בזה הדרך | ||
|- | |- | ||
| − | | | + | | |
| + | :We start from the large number and say: we divide 9 by 4; it is 2. We write it and we are left with 1. | ||
| + | |style="text-align:right;"|נתחי' מן המספר הגדול ונאמר נחלק ט' על ד' יהיו ב' ונכתבם וישאר בידינו א‫' | ||
|- | |- | ||
| − | | ||<span style="color:#0000FF>2</span>    ||2<span style="color:#0000FF>4</span>    | + | | |
| + | :We add this 1 to the 8 next to it; they are 18. | ||
| + | |style="text-align:right;"|ואותו הא' נחבר עם הח' הסמוך לו <s>וישאר י"ח</s> ויהיו י"ח | ||
| + | |- | ||
| + | | | ||
| + | :We say: we divide 18 by 4; it is 4. We write it and we are left with 2. | ||
| + | |style="text-align:right;"|ונאמר נחלק י"ח על ד' יהיו ד' ונכתבם וישארו בידינו ב‫' | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | :{| | ||
| + | |- | ||
| + | |<span style="color:red">9</span>8756||rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{red}{9\div4}}={\color{blue}{2}}+{\color{green}{r1}}}</math>||9<span style="color:red>8</span>756||rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{red}{{\color{green}{1}}8\div4}}={\color{blue}{4}}+{\color{green}{r2}}}</math>||98<span style="color:red>7</span>56 | ||
| + | |- | ||
| + | | ||<span style="color:#0000FF>2</span>    ||2<span style="color:#0000FF>4</span>    | ||
|- | |- | ||
|    <span style="color:red">4</span>||    <span style="color:red">4</span>||    <span style="color:red">4</span> | |    <span style="color:red">4</span>||    <span style="color:red">4</span>||    <span style="color:red">4</span> | ||
|} | |} | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | :::{| | + | :We add this 2 to the 7 that follows it; they are 27. |
| + | |style="text-align:right;"|ואלו הב' נחבר עם הז' הבא אחריו ויהיו כ"ז | ||
| + | |- | ||
| + | | | ||
| + | :We divide it by 4; it is 6. We write it and we are left with 3. | ||
| + | |style="text-align:right;"|ונחלקם על ד' ויהיו ו' ונכתבם וישארו בידינו ג‫' | ||
| + | |- | ||
| + | | | ||
| + | :We add this 3 to the [5] that follows it; they are 35. | ||
| + | |style="text-align:right;"|ואילו הג' נחבר עם הבא אחריו ויהיו ל"ה | ||
| + | |- | ||
| + | | | ||
| + | :We divide it by 4; it is 8. We write it and we are left with 3. | ||
| + | |style="text-align:right;"|ונחלקם על ד' יהיו ח' ונכתבם וישארו בידינו ג‫' | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | ::{| | ||
|- | |- | ||
|rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{red}{{\color{green}{2}}7\div4}}={\color{blue}{6}}+{\color{green}{r3}}}</math>||987<span style="color:red>5</span>6||rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{red}{{\color{green}{3}}5\div4}}={\color{blue}{8}}+{\color{green}{r3}}}</math>||9875<span style="color:red>6</span> | |rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{red}{{\color{green}{2}}7\div4}}={\color{blue}{6}}+{\color{green}{r3}}}</math>||987<span style="color:red>5</span>6||rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{red}{{\color{green}{3}}5\div4}}={\color{blue}{8}}+{\color{green}{r3}}}</math>||9875<span style="color:red>6</span> | ||
| Line 1,460: | Line 1,864: | ||
|    <span style="color:red">4</span>||    <span style="color:red">4</span> | |    <span style="color:red">4</span>||    <span style="color:red">4</span> | ||
|} | |} | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | ::::{| | + | :We add this 3 to the 6 that follows it; they are 36. |
| + | |style="text-align:right;"|ואילו הג' נחבר עם ו' הבא אחריו ויהיו ל"ו | ||
| + | |- | ||
| + | | | ||
| + | :We divide it by 4; it is 9. We write it. | ||
| + | |style="text-align:right;"|ונחלקם על ד' ויהיו ט' ונכתבם | ||
| + | |- | ||
| + | | | ||
| + | :Hence, we divide 98756 by 4 and the result is 24689. | ||
| + | |style="text-align:right;"|הרי שחלקנו צ"ח אלפים ותשנ"ו על ד' ועלו כ"ד אלפי' ות"רפ"ט | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | :::{| | ||
|- | |- | ||
|rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{red}{{\color{green}{3}}6\div4}}={\color{blue}{9}}}</math>||98756 | |rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{red}{{\color{green}{3}}6\div4}}={\color{blue}{9}}}</math>||98756 | ||
| Line 1,472: | Line 1,886: | ||
|    4 | |    4 | ||
|} | |} | ||
| − | |||
| − | |||
|- | |- | ||
| − | |If | + | |If we had 1, or 2, or 3 left at the end, they were parts of the 4 by which we divided the number. |
|style="text-align:right;"|ואם היה נשאר בידינו באחרונה א' או ב' או ג' היו חלקי' מד' שחלקנו עליו החשבון | |style="text-align:right;"|ואם היה נשאר בידינו באחרונה א' או ב' או ג' היו חלקי' מד' שחלקנו עליו החשבון | ||
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle8976\div5</math> | + | *If we want to divide the number 8976 by 5. |
| − | |style="text-align:right;"|וכן אלו רצינו לחלק החשבון ח' אלפים ותת"קע"ו על ה' | + | :<math>\scriptstyle8976\div5</math> |
| + | |style="text-align:right;"|וכן אלו רצינו לחלק החשבון ח' אלפים ותת"קע"ו על ה‫' | ||
|- | |- | ||
| | | | ||
| Line 1,497: | Line 1,910: | ||
|- | |- | ||
| | | | ||
| + | :We do this way: | ||
| + | |style="text-align:right;"|נעשה על זה הדרך | ||
| + | |- | ||
| + | | | ||
| + | :We say: divide 8 by 5; it is 1. We write it. 1 remains. | ||
| + | |style="text-align:right;"|ונאמר חלק ח' על ה' יהיה א' ונכתבנו ישאר ג‫' | ||
| + | |- | ||
| + | | | ||
| + | :We add this 3 to the 9 that follows it; they are 39. | ||
| + | |style="text-align:right;"|ואילו הג' נחבר עם הט' הבא אחריו ויהיו ל"ט | ||
| + | |- | ||
| + | | | ||
| + | :We divide it by 5; it is 7. We write it. 4 remains. | ||
| + | |style="text-align:right;"|נחלקם על ה' יהיו ז' ונכתבם וישארו ד‫' | ||
| + | |- | ||
| + | | colspan="2"| | ||
:{| | :{| | ||
|- | |- | ||
| Line 1,505: | Line 1,934: | ||
|   <span style="color:red">5</span>||   <span style="color:red">5</span>||   <span style="color:red">5</span> | |   <span style="color:red">5</span>||   <span style="color:red">5</span>||   <span style="color:red">5</span> | ||
|} | |} | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| + | :We add this 4 to the 7 that follows it; they are 47. | ||
| + | |style="text-align:right;"|ואלו הד' נחברם עם הז' הבא אחריו יהיו מ"ז | ||
| + | |- | ||
| + | | | ||
| + | :We divide it by 5; it is 9. We write it and we are left with 2. | ||
| + | |style="text-align:right;"|נחלקם על ה' ויהיו ט' ונכתבם וישארו בידינו ב‫' | ||
| + | |- | ||
| + | | | ||
| + | :We add this 2 to the 6 that follows it; they are 26. | ||
| + | |style="text-align:right;"|ואלו הב' נחברם עם ו' הבא אחריו ויהיו כ"ו | ||
| + | |- | ||
| + | | | ||
| + | :We divide it by 5; it is 5. We write it and we are left with 1. We write it above the 5, by which we divided the number. | ||
| + | |style="text-align:right;"|נחלקם על ה' ויהיו ה' ונכתבם וישאר בידינו א' ונכתבנו על ה' אשר חלקנו עליו החשבון | ||
| + | |- | ||
| + | | | ||
| + | :The result of the division mentioned is 1795 and a fifth. | ||
| + | |style="text-align:right;"|ויהי היוצא מן החלוקה הנזכר' אלף ות"שצ"ה וא' חומש | ||
| + | |- | ||
| + | | colspan="2"| | ||
::{| | ::{| | ||
|- | |- | ||
| Line 1,517: | Line 1,964: | ||
|   <span style="color:red">5</span>||   5 | |   <span style="color:red">5</span>||   5 | ||
|} | |} | ||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |If we want to divide a number by a number and the digits of the number you want to divide are smaller than the number by which you want to divide them, you should do this way: |
| − | |style="text-align:right;"|אמנם אילו רצינו לחלק מספר על מספר וחלקי המספר שאתה רוצה לחלק קטנים מהמספר שתרצה לחלקם בו אתה צריך לעשות על זה הדרך | + | |style="text-align:right;"|<big>אמנם</big> אילו רצינו לחלק מספר על מספר וחלקי המספר שאתה רוצה לחלק קטנים מהמספר שתרצה לחלקם בו אתה צריך לעשות על זה הדרך |
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle12345\div9</math> | + | *Example: we wish to divide 12345 by 9. |
| − | |style="text-align:right;"|דמיון זה רצינו לחלק י"ב אלפי' ושמ"ה על ט' | + | :<math>\scriptstyle12345\div9</math> |
| + | |style="text-align:right;"|<big>דמיון זה</big> רצינו לחלק י"ב אלפי' ושמ"ה על ט‫' | ||
| + | |- | ||
| + | | | ||
| + | | | ||
{|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | {|class="wikitable" style="margin-left: auto; margin-right: 0px; text-align:center;" | ||
|- | |- | ||
| Line 1,540: | Line 1,988: | ||
|- | |- | ||
| | | | ||
| − | : | + | :We say: divide 1 by 9; we cannot. |
| + | |style="text-align:right;"|אמרנו חלק א' על ט' לא נוכל‫<ref>נוכל: MS Ithaca illegible</ref> | ||
|- | |- | ||
| − | |<span style="color:red">12</span>345||rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{red}{12\div9}}={\color{blue}{1}}+{\color{green}{r3}}}</math>||12<span style="color:red>3</span>45||rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{red}{{\color{green}{3}}3\div9}}={\color{blue}{3}}+{\color{green}{r6}}}</math>||123<span style="color:red>4</span>5 | + | | |
| + | :We add 1 to the 2 that follows it; they are 12. | ||
| + | |style="text-align:right;"|נחבר א' עם הב' הבא אחריו ויהיו י"ב | ||
| + | |- | ||
| + | | | ||
| + | :Divide it by 9; it is 1. We write it and we are left with 3. | ||
| + | |style="text-align:right;"|חלקם על ט' יהיה א' ונכתבנו וישארו בידינו ג‫' | ||
| + | |- | ||
| + | | | ||
| + | :We add it to the 3 that follows it; they are 33. | ||
| + | |style="text-align:right;"|ונחברם עם הג' הבא אחריו ויהיו ל"ג | ||
| + | |- | ||
| + | | | ||
| + | :Divide it by 9; it is 3. We write it and we are left with 6. | ||
| + | |style="text-align:right;"|חלקם בט' ויהיו ג' ונכתבם וישארו בידינו ו‫' | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | :{| | ||
| + | |- | ||
| + | |<span style="color:red">12</span>345||rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{red}{12\div9}}={\color{blue}{1}}+{\color{green}{r3}}}</math>||12<span style="color:red>3</span>45||rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{red}{{\color{green}{3}}3\div9}}={\color{blue}{3}}+{\color{green}{r6}}}</math>||123<span style="color:red>4</span>5 | ||
|- | |- | ||
| || <span style="color:#0000FF>1</span>   || 1<span style="color:#0000FF>3</span>   | | || <span style="color:#0000FF>1</span>   || 1<span style="color:#0000FF>3</span>   | ||
| Line 1,548: | Line 2,016: | ||
|    <span style="color:red">9</span>||    <span style="color:red">9</span>||    <span style="color:red">9</span> | |    <span style="color:red">9</span>||    <span style="color:red">9</span>||    <span style="color:red">9</span> | ||
|} | |} | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| + | :We add this 6 to the 4 that follows it; they are 64. | ||
| + | |style="text-align:right;"|ונחבר אלו הו' עם הד' הבא אחריו ויהיו ס"ד | ||
| + | |- | ||
| + | | | ||
| + | :Divide it by 9; it is 7. We write it and we are left with 1. | ||
| + | |style="text-align:right;"|חלקם בט' <s>ו</s> ויהיו ז' ונכתבם וישאר בידינו <s>ו' ונחבר אילו הו'</s> א‫' | ||
| + | |- | ||
| + | | | ||
| + | :We add the 1 to the 5 that follows it; they are 15. | ||
| + | |style="text-align:right;"|נחבר הא' עם הה' הבא אחריו יהיו ט"ו | ||
| + | |- | ||
| + | | | ||
| + | :We say: divide 15 by 9; it is 1 and we are left with 6. We write it above the 9, by which we divided the whole number. | ||
| + | |style="text-align:right;"|ונאמר חלק ט"ו בט' יהיו א'‫<ref>א': MS Ithaca om.</ref> וישארו בידינו ו' ונכתוב אותם על‫<ref>ונכתוב אותם על: MS Ithaca ונחלקנו על ח'</ref> הט' אשר בו חלקנו כל‫<ref>כל: MS Ithaca om.</ref> החשבון | ||
| + | |- | ||
| + | | | ||
| + | :The result is that if we divide 12345 by 9, the result of division is 2371 and 6-ninths. | ||
| + | |style="text-align:right;"|והיוצא מזה הוא שאם נחלק י"ב אלפים וש'מ'ה' על ט' יהיה היוצא מן החלוקה‫<ref>יהיה היוצא מן החלוקה: MS Ithaca om.</ref> אלפים וש'ע'א' וו' תשיעיות | ||
| + | |- | ||
| + | | colspan="2"| | ||
:::{| | :::{| | ||
|- | |- | ||
| Line 1,560: | Line 2,046: | ||
|    <span style="color:red">9</span>||    9 | |    <span style="color:red">9</span>||    9 | ||
|} | |} | ||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| − | |'''Check''' | + | |<span style="color:green>'''Check:'''</span> If you want to check if our calculation is correct, we do as follows: |
| − | |style="text-align:right;"|ואם תרצה לבחון אם החשבון שעשינו הוא אמתי נעשה על זה הדרך | + | |style="text-align:right;"|ואם תרצה לבחון אם החשבון שעשינו הוא אמתי נעשה על זה הדרך |
| − | היינו שנקבץ המספרים המחולקים ונשליכם ט' ט' ומה שנשאר נחזיק בידינו | + | |- |
| − | אחר כן נקבץ מה שעלה בידינו בחלוק ונשליכם ט' ט' ומה שעלה בידינו נרבה אותו עם המחלק | + | |We sum up the digits of the dividend, cast out the nines and keep the remainder. |
| − | ומה שעלה בידינו מהרבוי נשליכם ט' ט' | + | |style="text-align:right;"|היינו שנקבץ המספרים המחולקים ונשליכם ט' ט' ומה שנשאר נחזיק בידינו |
| − | ואם החשבון הוא אמתי יעלה בידינו כמו שעלה בידינו בקבצנו המספרים המחולקים | + | |- |
| + | |Then, we sum up [the digits of] the result of division, cast out the nines and multiply what we received by the divisor. | ||
| + | |style="text-align:right;"|אחר כן נקבץ מה שעלה בידינו בחלוק ונשליכם ט' ט' ומה שעלה בידינו נרבה אותו עם המחלק | ||
| + | |- | ||
| + | |We cast out the nines from the product we received. | ||
| + | |style="text-align:right;"|ומה שעלה בידינו מהרבוי נשליכם ט' ט‫' | ||
| + | |- | ||
| + | |If the calculation is correct, we receive the same as what we received when we summed the digits of the dividend. | ||
| + | |style="text-align:right;"|ואם החשבון הוא אמתי יעלה בידינו כמו שעלה בידינו בקבצנו המספרים המחולקים | ||
|- | |- | ||
| | | | ||
| − | :: | + | *We will illustrate this in the calculation we wrote above: |
| + | :<math>\scriptstyle98756\div4</math> | ||
|style="text-align:right;"|וזה הדמיון נעשו בחשבון שכתבנו לעיל | |style="text-align:right;"|וזה הדמיון נעשו בחשבון שכתבנו לעיל | ||
|- | |- | ||
| Line 1,597: | Line 2,089: | ||
|- | |- | ||
| | | | ||
| − | :::<math>\scriptstyle{\color{blue}{9+8+7+5+6=35\longrightarrow35\equiv_98}}</math> | + | :First, we sum up the digits of the dividend and say: 6, 5, 7, 8, 9; the total is 35. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|היינו בתחלה נקבץ המספרי' המחולקים ונאמ' ו' וה' ז' וח' וט' בין כלם ל"ה |
| + | |- | ||
| + | | | ||
| + | :We cast out the nines; 8 remains. We write it next to the digits of the dividend. | ||
| + | :<math>\scriptstyle{\color{blue}{9+8+7+5+6=35\longrightarrow35\equiv_98}}</math> | ||
| + | |style="text-align:right;"|נשליכם ט' ט' ישארו ח' ונכת' ונכתבם אצל המספרי' המחולקים | ||
| + | |- | ||
| + | | | ||
| + | :Then, we sum up [the digits of] the result of division and say: 9, 8, 6, 4, 2; the total is 29. | ||
| + | |style="text-align:right;"|אחר כן נקבץ מה שעלה בחלוק ונאמ' ט' וח' וו' וד' ב' בין כלם כ"ט | ||
| + | |- | ||
| + | | | ||
| + | :We cast out the nines; 2 remains. We write it next to the result of division. | ||
| + | :<math>\scriptstyle{\color{blue}{2+4+6+8+9=29\longrightarrow29\equiv_92\longrightarrow2\times4=8}}</math> | ||
| + | |style="text-align:right;"|נשליכם ט' ט' וישארו ב' ונכתבם אצל מה שעלה בחלוק | ||
|- | |- | ||
| | | | ||
| − | :: | + | :Then, we multiply it by the divisor and say: 2 times 4 is 8. Therefore, we receive the same as we received when we summed the digits of the dividend, so the calculation is correct. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אחר כן נרבה אותו עם המחלק ונאמ' ב' פעמי' ד' ח' והנה עלה בידינו מה שעלה בקבצנו המספרי' המחולקים אם כן החשבון אמתי |
| − | אחר כן נרבה אותו עם המחלק ונאמ' ב' פעמי' ד' ח' והנה עלה בידינו מה שעלה בקבצנו המספרי' המחולקים אם כן החשבון אמתי | + | |- |
| + | |If 1, or 2, or 3 remains from the division [procedure], we do this way: | ||
| + | |style="text-align:right;"|ואם ישארו מן החלוק א' או ב' או ג' נעשה על זה הדרך | ||
|- | |- | ||
| − | | | + | |After we sum up [the digits of] the result of division and cast out the nines, we add [what remains] to what we have left [= to the remainder from the division]. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וזה שקבצנו אחר מה שעלה בחלוק והשלכנו אותם ט' ט' נקבצם עם מה שנשאר בידינו |
| − | וזה שקבצנו אחר מה שעלה בחלוק והשלכנו אותם ט' ט' נקבצם עם מה שנשאר בידינו | + | |- |
| − | ואם החשבון אמתי יעלה בידינו כמו שעלה בידינו בקבצנו המספרים המחולקים | + | |If the calculation is correct, what we receive is the same as what we received when we summed the digits of the dividend. |
| + | |style="text-align:right;"|ואם החשבון אמתי יעלה בידינו כמו שעלה בידינו בקבצנו המספרים המחולקים | ||
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle8976\div5</math> | + | *We will illustrate this in the second calculation we wrote above: |
| + | :<math>\scriptstyle8976\div5</math> | ||
|style="text-align:right;"|וזה הדמיון נעשו כחשבון השני שכתבנו למעלה | |style="text-align:right;"|וזה הדמיון נעשו כחשבון השני שכתבנו למעלה | ||
|- | |- | ||
| | | | ||
| − | :::<math>\scriptstyle{\color{blue}{8+9+7+6=30\longrightarrow30\equiv_93}}</math> | + | :First, we sum up the digits of the dividend and say: 6, 7, 8, 9; the total is 30. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|היינו בתחלה נקבץ המספרי' המחולקים ונאמר ו' וז' וח' וט' בין כלם ל‫' |
| + | |- | ||
| + | | | ||
| + | :We cast out the nines; we are left with 3. We write it next to the digits of the dividend. | ||
| + | :<math>\scriptstyle{\color{blue}{8+9+7+6=30\longrightarrow30\equiv_93}}</math> | ||
| + | |style="text-align:right;"|נשליכם ט' ט' וישאר בידינו ג' ונכתבנו אצל המספרי' המחולקים | ||
|- | |- | ||
| | | | ||
| Line 1,647: | Line 2,162: | ||
|- | |- | ||
| | | | ||
| − | :: | + | :Then, we sum up [the digits of] the result of division and say: 5, 9, 7, 1; the total is 22. |
| − | |style="text-align:right;"|אחר כן נקבץ מה שעלה בידינו בחלוק ונאמר ה' וט' וז' וא' בין כלם כ"ב | + | |style="text-align:right;"|אחר כן נקבץ מה שעלה בידינו בחלוק ונאמר ה' וט' וז' וא' בין כלם כ"ב |
| − | |||
| − | |||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | :We cast out the nines; we are left with 4. We write it next to the result of division. |
| + | |style="text-align:right;"|נשליכם ט' ט' וישאר בידינו ד' ונכתבנו אצל מה שעלה בחלוק | ||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | :Then, we multiply it by the divisor and say: 4 times 5 is 20. |
| + | |style="text-align:right;"|אחר כן נרבה אותו עם המחלק ונאמ' ד' פעמי' ה' הם כ‫' | ||
|- | |- | ||
| | | | ||
| − | *<math>\scriptstyle89765\div12</math> | + | :We cast out the nines; we are left with 2. We add it to the 1 we received from the division, which is written above the 5; they are 3. |
| − | |style="text-align:right;"|דמיון זה רצינו לחלק פ"ט אלפים ות"שס"ה על י"ב | + | :<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle1+7+9+5=22&\scriptstyle\longrightarrow22\equiv_94\longrightarrow4\times5=20\\&\scriptstyle\longrightarrow20\equiv_92\longrightarrow2+1=3\\\end{align}}}</math> |
| + | |style="text-align:right;"|ונשליכם ט'ט' וישאר בידינו ב' ונחבר עם הב' הא' שעלה בידינו מן החלוק הכתוב על ה' ויהיו ג‫' | ||
| + | |- | ||
| + | | | ||
| + | :Therefore, we receive the same as we received when we summed the digits [of the dividend], so the calculation is correct. | ||
| + | |style="text-align:right;"|הנה שעלה בידינו כמו שעלה בידינו בקבצנו המספרים אם כך הוא החשבון אמתי‫<ref>ואם תרצה לבחון... אם כך הוא החשבון אמתי: MS Ithaca om.</ref> | ||
| + | |- | ||
| + | !Another method of dividing a number by a number | ||
| + | |style="text-align:right;"|<big>דרך מספר על מספר בדרך אחרת</big> | ||
| + | |- | ||
| + | |If we want to divide a known number by another number and it is difficult for us to divide it by this number, we divide it twice and we receive as if we divided it once by that number. | ||
| + | |style="text-align:right;"|‫<ref>34v</ref>אם נרצה לחלק מספר ידוע על מספר אחר ויקשה עלינו לחלקו על המספר ההוא נחלק אותו ב' פעמים ויעלה בידינו כאלו חלקנוהו פעם אחת על המספר ההוא | ||
| + | |- | ||
| + | | | ||
| + | *Example: we wish to divide 89765 by 12. | ||
| + | :<math>\scriptstyle89765\div12</math> | ||
| + | |style="text-align:right;"|<big>דמיון זה</big> רצינו לחלק פ"ט אלפים ות"שס"ה על י"ב | ||
|- | |- | ||
| | | | ||
| Line 1,679: | Line 2,210: | ||
|- | |- | ||
| | | | ||
| − | : | + | :If we divide it by 12 once the result is 7480 and 5 parts of 12 and this is the order of the procedure: |
|style="text-align:right;"|והנה אם חלקנוה על י"ב בפעם אחת יעלה ז' אלפים ות"פ וה' חלקים מי"ב וזה סדר עשייתו | |style="text-align:right;"|והנה אם חלקנוה על י"ב בפעם אחת יעלה ז' אלפים ות"פ וה' חלקים מי"ב וזה סדר עשייתו | ||
|- | |- | ||
| Line 1,697: | Line 2,228: | ||
|- | |- | ||
| | | | ||
| − | : | + | :We say: divide 8 by 12; we cannot. |
| + | |style="text-align:right;"|אמרנו חלק ח' על י"ב לא נוכל | ||
|- | |- | ||
| − | | | + | | |
| + | :We add the 8 to the 9 that follows it; they are 89. | ||
| + | |style="text-align:right;"|נחבר אל ח' הט' הבא אחריו יהיו פ"ט | ||
|- | |- | ||
| − | | || <span style="color:#0000FF>7</span>   || 7<span style="color:#0000FF>4</span>   | + | | |
| + | :Divide it by 12; it is 7. We write it and we are left with 5. | ||
| + | |style="text-align:right;"|חלקם בי"ב יהיו ז' ונכתבם וישאר בידינו ה‫' | ||
| + | |- | ||
| + | | | ||
| + | :We add it to the 7 that follows it; they are 57. | ||
| + | |style="text-align:right;"|ונחבר אליה ז' הבא אחריו ויהיו נ"ז | ||
| + | |- | ||
| + | | | ||
| + | :Divide it by 12; it is 4. We write it and we are left with 9. | ||
| + | |style="text-align:right;"|חלקים בי"ב יהיו ד' ונכתבם וישארו בידינו ט‫' | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | :{| | ||
| + | |- | ||
| + | |<span style="color:red">89</span>765||rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{red}{89\div12}}={\color{blue}{7}}+{\color{green}{r5}}}</math>||89<span style="color:red>7</span>65||rowspan="3"|<math>\scriptstyle\xrightarrow{{\color{red}{{\color{green}{5}}7\div12}}={\color{blue}{4}}+{\color{green}{r9}}}</math>||897<span style="color:red>6</span>5 | ||
| + | |- | ||
| + | | || <span style="color:#0000FF>7</span>   || 7<span style="color:#0000FF>4</span>   | ||
|- | |- | ||
|   <span style="color:red">12</span>||   <span style="color:red">12</span>||   <span style="color:red">12</span> | |   <span style="color:red">12</span>||   <span style="color:red">12</span>||   <span style="color:red">12</span> | ||
|} | |} | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| + | :We add it to the 6 that follows it; they are 96. | ||
| + | |style="text-align:right;"|ונחבר אליהם ו' הבא אחריו יהיו צ"ו | ||
| + | |- | ||
| + | | | ||
| + | :Divide it by 12; it is 8. We write it. | ||
| + | |style="text-align:right;"|חלקים בי"ב יהיו ח' ונכתבם | ||
| + | |- | ||
| + | | | ||
| + | :We say: divide 5 by 12; we cannot. | ||
| + | |style="text-align:right;"|ונאמר חלק ה' על יב לא נוכל | ||
| + | |- | ||
| + | | | ||
| + | :Write a zero beneath the 5 and write the 5 above 12; it is 7780 and 5 parts of 12. | ||
| + | |style="text-align:right;"|ותכתוב ציפרא תחת הה' וה' תכתוב על י"ב יהיו ז' אלפים ותש"פ וה' חלקים מי"ב | ||
| + | |- | ||
| + | | colspan="2"| | ||
::::{| | ::::{| | ||
|- | |- | ||
| Line 1,717: | Line 2,282: | ||
|   <span style="color:red">12</span>||   12 | |   <span style="color:red">12</span>||   12 | ||
|} | |} | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :If we want to divide this same number in another way, we divide it by 3 once, then we divide the result by 4, so it is divided into 12 parts also. |
| − | + | :<math>\scriptstyle\left(89765\div3\right)\div4</math> | |
| − | |style="text-align:right;"|ואם נרצה לחלק זה החשבון בעצמו בעניין אחר נחלק אותו על ג' פעם אחת והעולה נחלק אותו על ד' ויהיה נחלק לי"ב ג"כ ונכתוב זה החשבון שנית ונחלקנו על ג' בתחילה ואחר זה נחלקנו על ד' | + | |style="text-align:right;"|ואם נרצה לחלק זה החשבון בעצמו בעניין אחר נחלק אותו על ג' פעם אחת והעולה נחלק אותו על ד' ויהיה נחלק לי"ב חלקים ג"כ |
| + | |- | ||
| + | | | ||
| + | :We write this number again and divide it first by 3, then we divide it by 4. | ||
| + | |style="text-align:right;"|ונכתוב זה החשבון שנית ונחלקנו על ג' בתחילה ואחר זה נחלקנו על ד‫' | ||
|- | |- | ||
| | | | ||
| Line 1,742: | Line 2,309: | ||
|- | |- | ||
| | | | ||
| + | :We say: divide 8 by 3; it is 2. We write it and 2 remains. | ||
| + | |style="text-align:right;"|ונאמר ח' חלק אותו על ג' יהיו ב' ונכתבם וישארו ב‫' | ||
| + | |- | ||
| + | | | ||
| + | :We add it to the 9 that follows it; they are 29. | ||
| + | |style="text-align:right;"|ונחבר‫<ref>ונחבר: MS Ithaca ונכתבם ונחבר</ref> אותם עם ט' הבא אחריו ויהיו כ"ט | ||
| + | |- | ||
| + | | | ||
| + | :Divide it by 3; it is 9. We write it and 2 remains. | ||
| + | |style="text-align:right;"|חלקם בג' יהיו ט' ונכתבם וישארו ב‫' | ||
| + | |- | ||
| + | | colspan="2"| | ||
:{| | :{| | ||
|- | |- | ||
| Line 1,750: | Line 2,329: | ||
|    <span style="color:red">3</span>||    <span style="color:red">3</span>||    <span style="color:red">3</span> | |    <span style="color:red">3</span>||    <span style="color:red">3</span>||    <span style="color:red">3</span> | ||
|} | |} | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| + | :We add it to the 7 that follows it; they are 27. | ||
| + | |style="text-align:right;"|ונחברם עם הז' הבא אחריו ויהיו כ"ז | ||
| + | |- | ||
| + | | | ||
| + | :We divide it by 3; it is 9. We write it. | ||
| + | |style="text-align:right;"|ונחלקם על ג' יהיו ט' ונכתבם | ||
| + | |- | ||
| + | | | ||
| + | :Then, we say: divide 6 by 3; it is 2. We write it. | ||
| + | |style="text-align:right;"|ונאמר אחר זה ו' חלק בג' יהיו ב' ונכתבם | ||
| + | |- | ||
| + | | | ||
| + | :Then, we say: divide 5 by 3; it is 1. We write it and 2 remains. We write it above the 3, by which we divided this number. | ||
| + | |style="text-align:right;"|ונאמר אחר זה ה' חלק בג' יהיו א' ונכתבנו וישארו ב' ונכתבם על הג' אשר בו חלקנו זה המספר | ||
| + | |- | ||
| + | | colspan="2"| | ||
::::{| | ::::{| | ||
|- | |- | ||
| Line 1,762: | Line 2,355: | ||
|    <span style="color:red">3</span>||    <span style="color:red">3</span>||    3 | |    <span style="color:red">3</span>||    <span style="color:red">3</span>||    3 | ||
|} | |} | ||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| + | :Then, we divide by 4 the number we received from the division and say: divide 2 by 4; we cannot. | ||
| + | |style="text-align:right;"|אחר זה נחלק זה המספר שעלה בידינו מן החלוקה על ד' ונאמר חלק ב' בד' לא נוכל | ||
| + | |- | ||
| + | | | ||
| + | :Add 2 to the 9 that follows it; they are 29. | ||
| + | |style="text-align:right;"|חבר ב' עם הט' הבא אחריו יהיו כ"ט | ||
| + | |- | ||
| + | | | ||
| + | :Divide it by 4; it is 7. We write it and we are left with 1. | ||
| + | |style="text-align:right;"|חלקם בד' יהיו ז' ונכתבם וישאר בידינו א'‫<ref>א': MS Ithaca ונכתוב א'</ref> | ||
| + | |- | ||
| + | | | ||
| + | :We add 1 to the 9 that follows it; they are 19. | ||
| + | |style="text-align:right;"|ונחבר א' עם הט' הבא אחריו יהיו י"ט | ||
| + | |- | ||
| + | | | ||
| + | :Divide it by 4; it is 4. We write it and we are left with 3. | ||
| + | |style="text-align:right;"|חלקם בד' יהיו ד' ונכתבם וישארו בידינו ג‫' | ||
| + | |- | ||
| + | | | ||
| + | :We add it to the 2 that follows it; they are 32. | ||
| + | |style="text-align:right;"|ונחברם עם הב' הבא אחריו ויהיו ל"ב | ||
| + | |- | ||
| + | | | ||
| + | :We divide it by 4; it is 8. We write it. | ||
| + | |style="text-align:right;"|ונחלקם בד' יהיו ח' ונכתבם | ||
| + | |- | ||
| + | | colspan="2"| | ||
:::{| | :::{| | ||
|- | |- | ||
| Line 1,777: | Line 2,395: | ||
|    <span style="color:red">4</span>||    <span style="color:red">4</span>||    4 | |    <span style="color:red">4</span>||    <span style="color:red">4</span>||    4 | ||
|} | |} | ||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| + | :We divide 1 by 4; we cannot. We write a zero beneath the 1 and we write the 1, which we cannot divide by 4, above the 4, by which we divide the number. | ||
| + | |style="text-align:right;"|ונחלק א' בד' ולא נוכל ונכתוב ציפרא‫<ref>ציפרא: MS Ithaca twice</ref> תחת הא' והא' שלא נוכל לחלקה בד' נכתוב אותה על הד' אשר עליה נחלק החשבון | ||
| + | |- | ||
| + | | colspan="2"| | ||
::::{| | ::::{| | ||
|- | |- | ||
| Line 1,792: | Line 2,411: | ||
|    4 | |    4 | ||
|} | |} | ||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :So, you have divided the number by 3 and 4 and it is as if you have divided it by 12. |
| − | |style="text-align:right;"|הרי שחלקת החשבון בג' וד' והוא כאלו חלקתו בי"ב | + | :<math>\scriptstyle{\color{blue}{\left(\frac{2}{3}\div4\right)+\frac{1}{4}=\frac{2+\left(3\sdot1\right)}{12}=\frac{2+3}{12}=\frac{5}{12}}}</math> |
| − | + | |style="text-align:right;"|הרי שחלקת החשבון בג' וד' והוא כאלו חלקתו בי"ב | |
| − | |||
|- | |- | ||
| − | | | + | | |
| − | + | :Multiply the 1 above the 4 by the 3 and say: 1 time 3 is 3; with the 2 above the 3, it is 5 and they are 5 parts of 12. | |
| + | |style="text-align:right;"|ותרבה אחר זה הא' שעל הד' עם הג' ואמור א' פעם ג' ג' וב' שעל הג' הרי ה' והם ה' חלקים מי"ב | ||
| + | |- | ||
| + | | | ||
| + | :It follows from this that if we first divide by 3, then we divide the result by 4 as we did, the result of division is 7480 and 5 parts of 12, as we received when we divided it once by 12 and this is clear. | ||
| + | |style="text-align:right;"|היוצא מדברינו שאם נחלק בראשונ' על ג' והיוצא נחלק על ד' על הדרך שעשינו יעלה מן החלוקה ז' אלפי' ות"פ וה' חלקים מי"ב כאשר עלה בידינו בעת שחלקנוהו פעם אחת על י"ב וזה מבואר | ||
|- | |- | ||
| | | | ||
| − | == | + | == The Method of Knowing the Numbers Summed One after the Other Successively == |
| − | + | |style="text-align:right;"|‫<ref>35r</ref><big>דרך ידיעת המספרים המקובצים זה אחר זה על</big> הסדר | |
|- | |- | ||
| | | | ||
| − | + | *{{#annot:1-10|669|QIjG}}If a person says: 1, 2, 3, 4, 5, 6, 7, 8, 9, 10 - how much is the total? | |
| + | :<math>\scriptstyle1+2+3+4+5+6+7+8+9+10=\sum_{i=1}^{10} i</math> | ||
|style="text-align:right;"|אם יאמר אדם א' וב' וג' וד' וה' וו' וז' וח' וטי' וי' כמה שוי בין כלם{{#annotend:QIjG}} | |style="text-align:right;"|אם יאמר אדם א' וב' וג' וד' וה' וו' וז' וח' וטי' וי' כמה שוי בין כלם{{#annotend:QIjG}} | ||
|- | |- | ||
| − | | | + | |You should understand, if the last term is an even number as this number or similar to it, always add one to the last term and multiply it by half the last term. |
| − | + | :<math>\scriptstyle{\color{OliveGreen}{\sum_{i=1}^{n} i=\left(n+1\right)\sdot\left(\frac{1}{2}\sdot n\right)}}</math> | |
| − | |style="text-align:right;"|יש לך להבין אם המספר האחרון הוא מספר זוג כמו זה מספר והדומה לו | + | |style="text-align:right;"|יש לך להבין אם המספר האחרון הוא מספר זוג כמו זה מספר והדומה לו תוסיף מספר אחד לעולם על הי' המספר האחרון ותרבה אותו על חצי המספר האחרו‫' |
|- | |- | ||
| | | | ||
| − | : | + | :Say: half 10 is 5. Multiply it by 11: 5 times 11 is 54 and so is [the sum of] the numbers mentioned. |
| − | + | :<math>\scriptstyle{\color{blue}{\sum_{i=1}^{10} i=\left(10+1\right)\sdot\left(\frac{1}{2}\sdot10\right)=11\sdot5=55}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואמור החצי מי' הוא ה' ותרבה אותו על י"א ה' פעמי' י"א נ"ה וכן יהיו המספרים הנזכרי‫' |
|- | |- | ||
| | | | ||
| − | + | *{{#annot:1-12|669|kTQb}}If he continues his question until 12. | |
| + | :<math>\scriptstyle\sum_{i=1}^{12} i</math> | ||
|style="text-align:right;"|ואם הלך בשאלתו עד י"ב{{#annotend:kTQb}} | |style="text-align:right;"|ואם הלך בשאלתו עד י"ב{{#annotend:kTQb}} | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\sum_{i=1}^{12} i=6\sdot13=78}}</math> | + | :Say: 6 times 13 is 78 and so is [their sum]. |
| − | |style="text-align:right;"|אמור ו' פעמי' י"ג ע"ח יהיו | + | :<math>\scriptstyle{\color{blue}{\sum_{i=1}^{12} i=6\sdot13=78}}</math> |
| + | |style="text-align:right;"|אמור ו'‫<ref>ו': MS Ithaca י'</ref> פעמי' י"ג ע"ח‫<ref>ע"ח: MS Ithaca וכן</ref> יהיו | ||
|- | |- | ||
| | | | ||
| − | + | *{{#annot:1-20|669|u3dY}}If he continues his question until 20. | |
| + | :<math>\scriptstyle\sum_{i=1}^{20} i</math> | ||
|style="text-align:right;"|ואם הלך בשאלתו עד כ‫'{{#annotend:u3dY}} | |style="text-align:right;"|ואם הלך בשאלתו עד כ‫'{{#annotend:u3dY}} | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\sum_{i=1}^{20} i=10\sdot21}}</math> | + | :Say: 10 times 21 and so is [their sum]. |
| + | :<math>\scriptstyle{\color{blue}{\sum_{i=1}^{20} i=10\sdot21}}</math> | ||
|style="text-align:right;"|אמור י' פעמים כ"א וכן יהיו | |style="text-align:right;"|אמור י' פעמים כ"א וכן יהיו | ||
|- | |- | ||
| | | | ||
| − | + | *{{#annot:1-100|669|YfUq}}If he continues his question until one hundred. | |
| + | :<math>\scriptstyle\sum_{i=1}^{100} i</math> | ||
|style="text-align:right;"|ואם הלך עד מאה{{#annotend:YfUq}} | |style="text-align:right;"|ואם הלך עד מאה{{#annotend:YfUq}} | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\sum_{i=1}^{100} i=50\sdot101}}</math> | + | :Say: 50 times 101 and so is [their sum]. |
| + | :<math>\scriptstyle{\color{blue}{\sum_{i=1}^{100} i=50\sdot101}}</math> | ||
|style="text-align:right;"|אמור נ' פעמים ק"א וכן יהיו | |style="text-align:right;"|אמור נ' פעמים ק"א וכן יהיו | ||
|- | |- | ||
| − | | | + | |Always calculate this way when the last term is even. |
|style="text-align:right;"|ואתה תחשוב לעולם בזה הדרך כשיהיה מספר האחרון זוג | |style="text-align:right;"|ואתה תחשוב לעולם בזה הדרך כשיהיה מספר האחרון זוג | ||
|- | |- | ||
| − | | | + | |If the last term is an odd number, multiply the greater half of this last term by the whole last term. |
| − | + | :<math>\scriptstyle{\color{OliveGreen}{\sum_{i=1}^{n} i=\left[\left(n+1\right)\sdot\frac{1}{2}\right]\sdot n}}</math> | |
| − | + | |style="text-align:right;"|ואם יהיה מספר האחרון מספר נפרד תרבה הרוב מהמספר ההוא האחרון על כל המספר האחרון | |
| − | |||
| − | |||
| − | |||
| − | |style="text-align:right;"|תרבה הרוב מהמספר ההוא האחרון על כל המספר האחרון | ||
|- | |- | ||
| | | | ||
| − | + | *{{#annot:1-7|669|Hvdz}}As the one who says: I continue my calculation until 7. How much is the total? | |
| + | :<math>\scriptstyle\sum_{i=1}^{7} i</math> | ||
|style="text-align:right;"|כגון האומר הלכתי בחשבוני עד ז' כמה יהיו כלם{{#annotend:Hvdz}} | |style="text-align:right;"|כגון האומר הלכתי בחשבוני עד ז' כמה יהיו כלם{{#annotend:Hvdz}} | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\sum_{i=1}^{7} i=\left[\left(7+1\right)\sdot\frac{1}{2}\right]\sdot7=4\sdot7=28}}</math> | + | :Say: the greater half of 7 is 4. Multiply it by 7; it is 28 and so is [their sum]. |
| − | |style="text-align:right;"|אמור הרוב מז' הם ד' תרבה אותם על ז' יהיו כ"ח וכן יהיו | + | :<math>\scriptstyle{\color{blue}{\sum_{i=1}^{7} i=\left[\left(7+1\right)\sdot\frac{1}{2}\right]\sdot7=4\sdot7=28}}</math> |
| + | |style="text-align:right;"|אמור הרוב מז' הם ד' תרבה אותם על <s>נ"ח</s> ז' יהיו כ"ח וכן יהיו | ||
|- | |- | ||
| | | | ||
| − | + | *{{#annot:1-9|669|2b1t}}If he continues his question until 9. | |
| + | :<math>\scriptstyle\sum_{i=1}^{9} i</math> | ||
|style="text-align:right;"|וכן אם הלך בשאלתו עד ט‫'{{#annotend:2b1t}} | |style="text-align:right;"|וכן אם הלך בשאלתו עד ט‫'{{#annotend:2b1t}} | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\sum_{i=1}^{9} i=\left[\left(9+1\right)\sdot\frac{1}{2}\right]\sdot9=5\sdot9=45}}</math> | + | :Say: the greater half of 9 is 5. Multiply it by 9; it is 45 and so is [their sum]. |
| + | :<math>\scriptstyle{\color{blue}{\sum_{i=1}^{9} i=\left[\left(9+1\right)\sdot\frac{1}{2}\right]\sdot9=5\sdot9=45}}</math> | ||
|style="text-align:right;"|אמור הרוב מט' הוא ה' מנה אותם בט' יהיו מ"ה וכן יהיו | |style="text-align:right;"|אמור הרוב מט' הוא ה' מנה אותם בט' יהיו מ"ה וכן יהיו | ||
|- | |- | ||
| | | | ||
| − | + | *{{#annot:1-17|669|T2rf}}If he continues until 17. | |
| + | :<math>\scriptstyle\sum_{i=1}^{17} i</math> | ||
|style="text-align:right;"|ואם הלך עד י"ז{{#annotend:T2rf}} | |style="text-align:right;"|ואם הלך עד י"ז{{#annotend:T2rf}} | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\sum_{i=1}^{17} i=9\sdot17}}</math> | + | :Multiply 9 by 17 and so is [their sum]. |
| − | |style="text-align:right;"|תרבה ט' על י"ז וכן יהיו | + | :<math>\scriptstyle{\color{blue}{\sum_{i=1}^{17} i=9\sdot17}}</math> |
| + | |style="text-align:right;"|תרבה ט' על‫<ref>על: MS Ithaca עד</ref> י"ז וכן יהיו | ||
|- | |- | ||
| | | | ||
| − | + | *{{#annot:1-19|669|CIoT}}If he continues until 19. | |
| + | :<math>\scriptstyle\sum_{i=1}^{19} i</math> | ||
|style="text-align:right;"|ואם הלך עד י"ט{{#annotend:CIoT}} | |style="text-align:right;"|ואם הלך עד י"ט{{#annotend:CIoT}} | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\sum_{i=1}^{19} i=10\sdot19}}</math> | + | :Multiply 10 by 19 and so is [their sum]. |
| − | |style="text-align:right;"|תרבה י' עם י"ט וכן יהיו | + | :<math>\scriptstyle{\color{blue}{\sum_{i=1}^{19} i=10\sdot19}}</math> |
| − | + | |style="text-align:right;"|תרבה י' עם י"ט וכן <s>ה</s> יהיו | |
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | :< | + | === <span style=color:green>Sum of Evens</span> === |
| + | |||
| | | | ||
| + | |- | ||
| + | |If he says: I summed all the even numbers successively. | ||
| + | |style="text-align:right;"|ואם יאמר קבצתי מספרים כלם זוגות על הסדר | ||
|- | |- | ||
| | | | ||
| − | + | *{{#annot:2-12|671|Gsfn}}As 2, 4, 6, 8, 10, 12 - how much is the total? | |
| + | :<math>\scriptstyle2+4+6+8+10+12=\sum_{i=1}^{6} 2i</math> | ||
|style="text-align:right;"|כמו ב' וד' וו' וח' וי' וי"ב כמה יהיה בין כלם{{#annotend:Gsfn}} | |style="text-align:right;"|כמו ב' וד' וו' וח' וי' וי"ב כמה יהיה בין כלם{{#annotend:Gsfn}} | ||
| + | |- | ||
| + | |Multiply half the last term by the number that follows this half. | ||
| + | |style="text-align:right;"|תרבה החצי מן המספר האחרון על המספר אשר אחרי החצי ההוא | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\sum_{i=1}^{n} 2i=\left(\frac{1}{2}\sdot2n\right)\sdot\left[\left(\frac{1}{2}\sdot2n\right)+1\right]}}</math> | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{ | + | :Say: half 12 is 6 and the number that follows 6 is 7. 6 times 7 is 42 and so is [their sum]. |
| − | + | |style="text-align:right;"|ואמור החצי מי"ב הוא ו' והמספר שהוא אחר ו' הוא ז' ו' פעמים ז' הם <s>י"ב</s> מ"ב וכן יהיו | |
| + | |- | ||
| + | | colspan="2"| | ||
| + | :<math>\scriptstyle{\color{blue}{2+4+6+8+10+12=\sum_{i=1}^{6} 2i=\left(\frac{1}{2}\sdot12\right)\sdot\left[\left(\frac{1}{2}\sdot12\right)+1\right]=6\sdot\left(6+1\right)=6\sdot7=42}}</math> | ||
|- | |- | ||
| | | | ||
| − | + | *{{#annot:2-20|671|qYPE}}If he says: I continued until twenty. | |
| + | :<math>\scriptstyle2+\ldots+20=\sum_{i=1}^{10} 2i</math> | ||
|style="text-align:right;"|ואם אומר הלכתי עד עשרי‫'{{#annotend:qYPE}} | |style="text-align:right;"|ואם אומר הלכתי עד עשרי‫'{{#annotend:qYPE}} | ||
|- | |- | ||
| | | | ||
| − | :: | + | :Say: half twenty is 10 and the number that follows 10 is 11. 10 times [11] is 110 and so is [their sum]. |
|style="text-align:right;"|אמור החצי מעשרי' הוא י' והמספר שאחר י' הוא י"א י' פעמים הם ק"י וכן יהיו | |style="text-align:right;"|אמור החצי מעשרי' הוא י' והמספר שאחר י' הוא י"א י' פעמים הם ק"י וכן יהיו | ||
|- | |- | ||
| − | | | + | | colspan="2"| |
| + | :<math>\scriptstyle{\color{blue}{2+\ldots+20=\sum_{i=1}^{10} 2i=\left(\frac{1}{2}\sdot20\right)\sdot\left[\left(\frac{1}{2}\sdot20\right)+1\right]=10\sdot\left(10+1\right)=10\sdot11=110}}</math> | ||
| + | |- | ||
| + | |Always calculate this way. | ||
|style="text-align:right;"|ועל זה הדרך תחשוב לעולם | |style="text-align:right;"|ועל זה הדרך תחשוב לעולם | ||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | :< | + | === <span style=color:green>Sum of Odds</span> === |
| | | | ||
| + | |- | ||
| + | |If he says: I summed all the odd numbers successively. | ||
| + | |style="text-align:right;"|ואם יאמר קבצתי מספרים כלם נפרדי' על הסדר | ||
|- | |- | ||
| | | | ||
| − | + | *{{#annot:1-9|670|rGAn}}As 1, 3, 5, 7, 9 - how much is the total? | |
| + | :<math>\scriptstyle1+3+5+7+9=\sum_{i=1}^{5} \left(2i-1\right)</math> | ||
|style="text-align:right;"|כגון א' וג' וה' וז' וט' כמה הם בין כלם{{#annotend:rGAn}} | |style="text-align:right;"|כגון א' וג' וה' וז' וט' כמה הם בין כלם{{#annotend:rGAn}} | ||
| + | |- | ||
| + | |Take the greater half of the last term and multiply it by itself. | ||
| + | |style="text-align:right;"|קח הרוב מהמספר האחרון ותרבה הרוב בעצמו | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\sum_{i=1}^{n} \left(2i-1\right)=\left[\left[\left(2n-1\right)+1\right]\sdot\frac{1}{2}\right]^2}}</math> | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{1+3+5+7+9=\sum_{i=1}^{5} \left(2i-1\right)=\left[\left(9+1\right)\sdot\frac{1}{2}\right]^2=5^2=25}}</math> | + | :Say: the greater half of 9 is 5. 5 times 5 is 25 and so is [their sum]. |
| − | + | |style="text-align:right;"|ואמור הרוב מט' הוא ה' וה' פעמים ה' הוא כ"ה וכן יהיו כלם | |
| + | |- | ||
| + | | colspan="2"| | ||
| + | :<math>\scriptstyle{\color{blue}{1+3+5+7+9=\sum_{i=1}^{5} \left(2i-1\right)=\left[\left(9+1\right)\sdot\frac{1}{2}\right]^2=5^2=25}}</math> | ||
|- | |- | ||
| | | | ||
| − | + | *{{#annot:1-11|670|NxSy}}If he continues his question until 11. | |
| + | :<math>\scriptstyle1+\ldots+11=\sum_{i=1}^{6} \left(2i-1\right)</math> | ||
|style="text-align:right;"|ואם הלך בשאלתו עד י"א{{#annotend:NxSy}} | |style="text-align:right;"|ואם הלך בשאלתו עד י"א{{#annotend:NxSy}} | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{1+\ldots+11=\sum_{i=1}^{6} \left(2i-1\right)=\left[\left(11+1\right)\sdot\frac{1}{2}\right]^2=6^2}}</math> | + | :Say: 6 times 6 and so is [their sum]. |
| + | :<math>\scriptstyle{\color{blue}{1+\ldots+11=\sum_{i=1}^{6} \left(2i-1\right)=\left[\left(11+1\right)\sdot\frac{1}{2}\right]^2=6^2}}</math> | ||
|style="text-align:right;"|אמור ו' פעמי' ו' וכן יהיו | |style="text-align:right;"|אמור ו' פעמי' ו' וכן יהיו | ||
|- | |- | ||
| | | | ||
| − | + | *{{#annot:1-13|670|2g6v}}If he continues until 13. | |
| + | :<math>\scriptstyle1+\ldots+13=\sum_{i=1}^{7} \left(2i-1\right)</math> | ||
|style="text-align:right;"|ואם הלך עד י"ג{{#annotend:2g6v}} | |style="text-align:right;"|ואם הלך עד י"ג{{#annotend:2g6v}} | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{1+\ldots+13=\sum_{i=1}^{7} \left(2i-1\right)=\left[\left(13+1\right)\sdot\frac{1}{2}\right]^2=7^2}}</math> | + | :Say: 7 times 7 and so is [their sum]. |
| + | :<math>\scriptstyle{\color{blue}{1+\ldots+13=\sum_{i=1}^{7} \left(2i-1\right)=\left[\left(13+1\right)\sdot\frac{1}{2}\right]^2=7^2}}</math> | ||
|style="text-align:right;"|אמור ז' פעמי' ז' וכן יהיו | |style="text-align:right;"|אמור ז' פעמי' ז' וכן יהיו | ||
|- | |- | ||
| + | |Always calculate this way. | ||
| + | |style="text-align:right;"|ועל זה הדרך תחשוב לעולם | ||
| + | |- | ||
| + | | | ||
| + | === <span style=color:green>Sum of Powers of Two</span> === | ||
| + | |||
| | | | ||
| − | |||
|- | |- | ||
| − | + | |If a man says: I summed the doubles to each other. | |
| − | + | :<math>\scriptstyle\sum_{i=m}^{n} 2^i</math> | |
| + | |style="text-align:right;"|ואם יאמר אדם קבצתי מספרים כפולי' זה על זה | ||
|- | |- | ||
| | | | ||
| − | + | *{{#annot:1-32|674|y2at}}As 1, 2, 4, 8, 16, 32. | |
| + | :<math>\scriptstyle1+2+4+8+16+32=\sum_{i=1}^{6} 2^{i-1}</math> | ||
|style="text-align:right;"|כגון א' וב' וד' וח' וי"ו ול"ב{{#annotend:y2at}} | |style="text-align:right;"|כגון א' וב' וד' וח' וי"ו ול"ב{{#annotend:y2at}} | ||
|- | |- | ||
| − | | | + | |He starts with whichever [number] he wants to start and ends with whichever number he wants to end: double the last term and subtract the first term. |
| − | :<math>\scriptstyle\sum_{i=m}^{n} 2^i=\left(2\sdot2^n\right)-2^m</math> | + | :<math>\scriptstyle{\color{OliveGreen}{\sum_{i=m}^{n} 2^i=\left(2\sdot2^n\right)-2^m}}</math> |
|style="text-align:right;"|בכל מקום שירצה להתחיל יתחיל ובכל חשבון שיחפוץ לסיים יסיים כפול המספר האחרון וחסר המספר הראשון | |style="text-align:right;"|בכל מקום שירצה להתחיל יתחיל ובכל חשבון שיחפוץ לסיים יסיים כפול המספר האחרון וחסר המספר הראשון | ||
|- | |- | ||
| | | | ||
| − | + | *{{#annot:1-32|674|6kg5}}Example: one says: I summed the doubles from 1 to 32. How much is their sum? | |
| − | |style="text-align:right;"|דמיון זה האומר קבצתי מספרים כפולי' מא' ועד ל"ב כמה הם{{#annotend:6kg5}} | + | :<math>\scriptstyle1+\ldots+32=\sum_{i=1}^{6} 2^{i-1}</math> |
| + | |style="text-align:right;"|<big>דמיון זה</big> האומר קבצתי מספרים כפולי' מא' ועד ל"ב כמה הם{{#annotend:6kg5}} | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{1+\ldots+32=\sum_{i=1}^{6} 2^{i-1}=\left(2\sdot32\right)-1=64-1=63}}</math> | + | :Double 32, which is the last term; it is 64. Subtract the first term, which is 1; 63 remains and so is [their sum]. |
| − | |style="text-align:right;"|ואתה כפול ל"ב שהוא המספר האחרון ויהיו ס"ד וחסר המספר הראשון שהוא א' וישארו ס"ג וכן יהיו | + | :<math>\scriptstyle{\color{blue}{1+\ldots+32=\sum_{i=1}^{6} 2^{i-1}=\left(2\sdot32\right)-1=64-1=63}}</math> |
| + | |style="text-align:right;"|ואתה כפול ל"ב שהוא המספר האחרון ויהיו ס"ד וחסר המספר הראשון שהוא א' וישארו ס"ג‫<ref>ס"ג: MS Ithaca ג'</ref> וכן יהיו | ||
|- | |- | ||
| | | | ||
| − | + | *{{#annot:1-64|674|WJGn}}If he says: I started from 1 and doubled until 64. How much is [their sum]? | |
| − | |style="text-align:right;"|ואם יאמ' התחלתי מא' וכפלתי עד ס"ד כמה יהיו{{#annotend:WJGn}} | + | :<math>\scriptstyle1+\ldots+64=\sum_{i=1}^{7} 2^{i-1}</math> |
| + | |style="text-align:right;"|ואם יאמ' התחלתי מא' וכפלתי עד ס"ד‫<ref>ס"ד: MS Ithaca קכ"ח</ref> כמה יהיו{{#annotend:WJGn}} | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{1+\ldots+64=\sum_{i=1}^{7} 2^{i-1}=\left(2\sdot64\right)-1=128-1=127}}</math> | + | :Double 64; it is 128. Subtract one; it is 127. |
| − | + | |style="text-align:right;"|כפול <s>קכ"ח</s> ס"ד יהיו קכ"ח חסר אחת יהיו קכ"ז | |
| + | |- | ||
| + | | colspan="2"| | ||
| + | :<math>\scriptstyle{\color{blue}{1+\ldots+64=\sum_{i=1}^{7} 2^{i-1}=\left(2\sdot64\right)-1=128-1=127}}</math> | ||
|- | |- | ||
| | | | ||
| − | + | *{{#annot:1-128|674|IUR1}}If he says: I started from one and doubled until 128. How much is [their sum]? | |
| − | |style="text-align:right;"|ואם יאמר התחלתי מאחד וכפלתי עד קכ"ח כמה יהיו{{#annotend:IUR1}} | + | :<math>\scriptstyle1+\ldots+128=\sum_{i=1}^{8} 2^{i-1}</math> |
| + | |style="text-align:right;"|ואם יאמר התחלתי מאחד וכפלתי עד <s>ס"ד</s> קכ"ח כמה יהיו{{#annotend:IUR1}} | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{1+\ldots+128=\sum_{i=1}^{8} 2^{i-1}=\left(2\sdot128\right)-1=256-1=255}}</math> | + | :Double 128; it is 256. Subtract 1; it is 255. |
| − | + | |style="text-align:right;"|כפול קכ"ח יהיו רנ"ו חסר א' יהיה רנ"ה | |
| + | |- | ||
| + | | colspan="2"| | ||
| + | :<math>\scriptstyle{\color{blue}{1+\ldots+128=\sum_{i=1}^{8} 2^{i-1}=\left(2\sdot128\right)-1=256-1=255}}</math> | ||
|- | |- | ||
| | | | ||
| − | + | *{{#annot:4-32|674|ghpP}}If he says: I started from 4 and doubled until 32. How much is their sum? | |
| + | :<math>\scriptstyle4+\ldots+32=\sum_{i=3}^{6} 2^{i-1}</math> | ||
|style="text-align:right;"|ואם יאמר התחלתי מד' וכפלתי עד ל"ב כמה יהיו{{#annotend:ghpP}} | |style="text-align:right;"|ואם יאמר התחלתי מד' וכפלתי עד ל"ב כמה יהיו{{#annotend:ghpP}} | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{4+\ldots+32=\sum_{i=3}^{6} 2^{i-1}=\left(2\sdot32\right)-4=64-4=60}}</math> | + | :Double 32; it is 64. Subtract 4; it is 60. |
| − | |style="text-align:right;"|כפול ל"ב | + | :<math>\scriptstyle{\color{blue}{4+\ldots+32=\sum_{i=3}^{6} 2^{i-1}=\left(2\sdot32\right)-4=64-4=60}}</math> |
| + | |style="text-align:right;"|כפול ל"ב יהיה ס"ד חסר ד' יהיו ס‫' | ||
|- | |- | ||
| + | |The procedure is to double the last and subtract the first. | ||
| + | |style="text-align:right;"|והחשבון המסור לזה לכפול הא <s>ל</s> האחרון ולחסר הראשון | ||
| + | |- | ||
| + | | | ||
| + | === <span style=color:green>Sum of Powers of Three</span> === | ||
| + | |||
| | | | ||
| − | |||
|- | |- | ||
| − | + | |If a man asks about [the sum of] the triples to each other. | |
| − | + | :<math>\scriptstyle\sum_{i=m}^{n} 3^i</math> | |
| + | |style="text-align:right;"|‫<ref>35v</ref><big>ואם ישאל</big> אדם על מספרי' משולשים זה על זה | ||
|- | |- | ||
| | | | ||
| − | + | *{{#annot:1-27|675|mIQm}}As 1, 3, 9, 27. | |
| + | :<math>\scriptstyle1+3+9+27=\sum_{i=1}^{4} 3^{i-1}</math> | ||
|style="text-align:right;"|כגון א' ג' ט' כ"ז{{#annotend:mIQm}} | |style="text-align:right;"|כגון א' ג' ט' כ"ז{{#annotend:mIQm}} | ||
|- | |- | ||
| − | | | + | |He adds to the last its half after subtracting the first, with which he starts, then adds the first to it. |
| − | + | |style="text-align:right;"|יוסיף על האחרון <s>כ"ז</s> חציו אחר גרעון הראשון שהתחיל בו ועוד יוסיף הראשון עמו | |
| − | |||
| − | |style="text-align:right;"|יוסיף על האחרון כ"ז חציו אחר גרעון הראשון שהתחיל בו ועוד יוסיף הראשון עמו | ||
|- | |- | ||
| − | + | | colspan="2"| | |
| − | + | :<math>\scriptstyle{\color{OliveGreen}{\sum_{i=m}^{n} 3^i=\left(3^n-3^m\right)+\left[\frac{1}{2}\sdot\left(3^n-3^m\right)\right]+3^m}}</math> | |
|- | |- | ||
| | | | ||
| − | : | + | :He subtracts the first term, which is 1, from the last term, which is 27; 26 remains. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|דהיינו שיגרע מכ"ז שהוא המספר האחרון המספר הראשון שהוא א' ישארו כ"ו |
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle\sum_{i= | + | :He says: 26 plus its half, which is 13, is 39. With 1, which is the first term, it is 40 and this is the [sum of] 1, 3, 9, 27. |
| − | + | |style="text-align:right;"|יאמר כ"ו וחציו שהוא י"ג הם ל"ט וא' שהוא החשבון הראשון הרי מ' וכן הם א'ג'ט' כ"ז | |
| + | |- | ||
| + | | colspan="2"| | ||
| + | :<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle1+3+9+27&\scriptstyle=\sum_{i=1}^{4} 3^{i-1}=\left(27-1\right)+\left[\frac{1}{2}\sdot\left(27-1\right)\right]+1\\&\scriptstyle=26+\left(\frac{1}{2}\sdot26\right)+1=26+13+1=39+1=40\\\end{align}}}</math> | ||
|- | |- | ||
| | | | ||
| − | + | === <span style=color:green>Sum of Powers of Four</span> === | |
| − | | | + | |
| + | | | ||
|- | |- | ||
| − | | | + | |If he asks about the quadruples. |
| − | + | :<math>\scriptstyle\sum_{i=m}^{n} 4^i</math> | |
| − | + | |style="text-align:right;"|<big>ואם ישאל</big> על מספרי מרובעים | |
| − | |||
| − | |||
| − | |style="text-align:right;"| | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | === | + | *{{#annot:1-16|676|aAn8}}As 1, 4, 16. |
| − | + | :<math>\scriptstyle1+4+16=\sum_{i=1}^{3} 4^{i-1}</math> | |
| + | |style="text-align:right;"|כגון א' ד' י"ו{{#annotend:aAn8}} | ||
| + | |- | ||
| + | |He adds to the last its third after subtracting the first, then adds the first to it. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\sum_{i=m}^{n} 4^i=\left(4^n-4^m\right)+\left[\frac{1}{3}\sdot\left(4^n-4^m\right)\right]+4^m}}</math> | ||
| + | |style="text-align:right;"|יוסיף על האחרון שליש אחר גרעון הראשון ועוד יוסיף הראשון עמו | ||
| + | |- | ||
| | | | ||
| + | :Example: 1, 4, 16 are 21. | ||
| + | |style="text-align:right;"|<big>דמיון זה</big> א' ד' י"ו הם כ"א | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:two men|657|hue5}}A man is walking ten miles a day. | + | :The last term is 16. Subtract the first term, which is 1; 15 remains. |
| − | :Another man is walking one mile on the first day, two miles on the second day, three miles on the third day, four miles on the fourth day, and so on he goes on walking in each day [one mile more]. | + | |style="text-align:right;"|המספר האחרון הוא י"ו חסר המספר הראשון א שהוא א' ישארו ט"ו |
| − | :In how many days will [ | + | |- |
| + | | | ||
| + | :Add to 15 its third, which is 5; it is 20. Add to it [the first] term; it is 21. | ||
| + | |style="text-align:right;"|הוסף על ט"ו שלישו שהוא ה' יהיו כ' הוסף עליהם המספר יהיו כ"א | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | :<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle1+4+16&\scriptstyle=\sum_{i=1}^{3} 4^{i-1}=\left(16-1\right)+\left[\frac{1}{3}\sdot\left(16-1\right)\right]+1\\&\scriptstyle=15+\left(\frac{1}{3}\sdot15\right)+1=15+5+1=20+1=21\\\end{align}}}</math> | ||
| + | |- | ||
| + | |And so on. | ||
| + | |style="text-align:right;"|וכן לעולם | ||
| + | |- | ||
| + | |The rule: | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\sum_{i=m}^{n} a^i=\left(a^n-a^m\right)+\left[\frac{1}{a-1}\sdot\left(a^n-a^m\right)\right]+a^m}}</math> | ||
| + | |style="text-align:right;"|והכלל המסור לזה | ||
| + | |- | ||
| + | |For the triples he adds a half. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\sum_{i=m}^{n} 3^i=\left(a^n-a^m\right)+\left[\frac{1}{2}\sdot\left(a^n-a^m\right)\right]+a^m}}</math> | ||
| + | |style="text-align:right;"|למשולש יוסיף החצי | ||
| + | |- | ||
| + | |For the quadruples he adds a third. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\sum_{i=m}^{n} 4^i=\left(a^n-a^m\right)+\left[\frac{1}{3}\sdot\left(a^n-a^m\right)\right]+a^m}}</math> | ||
| + | |style="text-align:right;"|ולמרובע יוסיף השלישי | ||
| + | |- | ||
| + | |For the quintuples he adds a quarter. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\sum_{i=m}^{n} 5^i=\left(a^n-a^m\right)+\left[\frac{1}{4}\sdot\left(a^n-a^m\right)\right]+a^m}}</math> | ||
| + | |style="text-align:right;"|ולמחומש יוסיף <s>ר</s> הרביע | ||
| + | |- | ||
| + | |For the sextuples he adds a fifth. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{\sum_{i=m}^{n} 6^i=\left(a^n-a^m\right)+\left[\frac{1}{5}\sdot\left(a^n-a^m\right)\right]+a^m}}</math> | ||
| + | |style="text-align:right;"|ולמשושה יוסיף החומש | ||
| + | |- | ||
| + | |It is always done this way. | ||
| + | |style="text-align:right;"|ועל הדרך הזה יעשה לעולם | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | === <span style=color:green>Motion Problems - Pursuit</span> === | ||
| + | |||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:two men|657|hue5}}If it is said: A man is walking ten miles a day. | ||
| + | :Another man is walking one mile on the first day, two miles on the second day, three miles on the third day, four miles on the fourth day, and so on he goes on walking in each day [one mile more]. | ||
| + | :In how many days will they walk the same [total distance]? | ||
:<math>\scriptstyle10X=\sum_{i=1}^x i</math> | :<math>\scriptstyle10X=\sum_{i=1}^x i</math> | ||
| − | |style="text-align:right;"|אם יאמר אדם אחד הולך בכל יום ויום י' מילי‫'<br> | + | |style="text-align:right;"|<big>אם יאמר אדם</big> אחד הולך בכל יום ויום י' מילי‫'<br> |
ואדם אחר הולך ביום אחד א' מיל וביום ב' ב' מילי' וביום ג' ג' מילים וביום ד' ד' מילי' וכן מוסיף והולך בכל יום ויום<br> | ואדם אחר הולך ביום אחד א' מיל וביום ב' ב' מילי' וביום ג' ג' מילים וביום ד' ד' מילי' וכן מוסיף והולך בכל יום ויום<br> | ||
כמה ימים יעמדו בשוה{{#annotend:hue5}} | כמה ימים יעמדו בשוה{{#annotend:hue5}} | ||
|- | |- | ||
| | | | ||
| − | : | + | :Double the 10 miles that the one who walks 10 miles every day walks; it is 20. |
| − | |style="text-align:right;"|אתה כפול הי' מילי' שמהלך אותו שמהלך בכל יום ויום י' מילין ויהיו כ‫' | + | |style="text-align:right;"|אתה כפול הי' מילי' שמהלך אותו שמהלך בכל יום ויום י' מילין ויהיו כ‫' |
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :Subtract 1 from it; 19 remains. |
| − | + | :<math>\scriptstyle{\color{blue}{x=\left(2\sdot10\right)-1=20-1=19}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|תפחות מהם א' ישארו י"ט |
|- | |- | ||
| | | | ||
| − | ::the one who adds another mile each day walks in 19 days: <math>\scriptstyle{\color{blue}{1+\ldots+19=\left[\left(19+1\right)\sdot\frac{1}{2}\right]\sdot19=10\sdot19=190}}</math> | + | :We find that in 19 [days] they walk the same [total distance]. |
| + | |style="text-align:right;"|נמצא שבי"ט יעמדו בשוה | ||
| + | |- | ||
| + | | | ||
| + | :<span style=color:green>'''Check:'''</span> | ||
| + | :The one who walks 10 miles a day walks in 19 days 190 miles, as the product of 10 by 19, which is 190. | ||
| + | :<math>\scriptstyle{\color{blue}{10\sdot19=190}}</math> | ||
| + | |style="text-align:right;"|שצד אותו שמהלך בכל יום י' מילי' בי"ט ימים יהלך ק"צ מילי' כמניין י' על י"ט שהם ק"צ | ||
| + | |- | ||
| + | | | ||
| + | :The one who adds another mile each day walks in 19 days 190 miles also: take the greater half of 19; it is 10. Multiply it by 19; it is 190. | ||
| + | :<math>\scriptstyle{\color{blue}{1+\ldots+19=\left[\left(19+1\right)\sdot\frac{1}{2}\right]\sdot19=10\sdot19=190}}</math> | ||
|style="text-align:right;"|ואותו שמוסיף בכל יום מיל אחד בי"ט ימים יהלך ג"כ ק"צ מילין<br> | |style="text-align:right;"|ואותו שמוסיף בכל יום מיל אחד בי"ט ימים יהלך ג"כ ק"צ מילין<br> | ||
| − | כיצד תפוש הרוב מי"ט והם י' ותכפול אותו על י"ט | + | כיצד תפוש הרוב מי"ט והם י' ותכפול אותו על י"ט ויהיה ק"צ |
|- | |- | ||
| − | | | + | |Always double the [number] of miles that the one who walks the same distance [each day] walks, then subtract one from it and this is the number of days, in which they walk the same [total distance]. |
| − | + | :<math>\scriptstyle{\color{OliveGreen}{ax=\sum_{i=1}^x i\longrightarrow x=2a-1}}</math> | |
| − | :<math>\scriptstyle ax=\sum_{i=1}^x i\longrightarrow x=2a-1</math> | ||
|style="text-align:right;"|וכן לעולם תכפול המילי' שמהלך המילי' שמהלך הקבוע ותפחות מהם אחד וכמספרם יהיו הימים שיעמדו בשוה | |style="text-align:right;"|וכן לעולם תכפול המילי' שמהלך המילי' שמהלך הקבוע ותפחות מהם אחד וכמספרם יהיו הימים שיעמדו בשוה | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:two men|657|54en}}A man is walking ten miles a day. | + | *{{#annot:two men|657|54en}}If it is said: A man is walking ten miles a day. |
:Another man is walking two miles on the first day, four [miles] on the second day, six [miles] on the third day, eight [miles] on the fourth day, and so on he adds two [miles more each day]. | :Another man is walking two miles on the first day, four [miles] on the second day, six [miles] on the third day, eight [miles] on the fourth day, and so on he adds two [miles more each day]. | ||
| − | :In how many days will [ | + | :In how many days will they walk the same [total distance]? |
:<math>\scriptstyle10X=\sum_{i=1}^x 2i</math> | :<math>\scriptstyle10X=\sum_{i=1}^x 2i</math> | ||
| − | |style="text-align:right;"|ואם יאמר אדם אחד הולך בכל יום י' מילי‫'<br> | + | |style="text-align:right;"|<big>ואם יאמר</big> אדם אחד הולך בכל יום י' מילי‫'<br> |
| − | ואדם אחר הולך ביום אחד ב' מילין וביום שני ד' וביום שלישי ו' וביום רביעי ח' וכן לעולם הוא מוסיף ב' והולך על הסדר<br> | + | ואדם‫<ref>אחד הולך... ואדם: MS Ithaca twice</ref> אחר הולך ביום אחד ב' מילין וביום שני ד' וביום שלישי ו' וביום רביעי ח' וכן לעולם הוא מוסיף ב' והולך על הסדר<br> |
בכמה ימים יעמדו בשוה{{#annotend:54en}} | בכמה ימים יעמדו בשוה{{#annotend:54en}} | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{x=10-1=9}}</math> | + | :Subtract 1 from the miles that the one who walks the same distance every day walks; 9 remains. |
| − | |style="text-align:right;"|אתה תפחות אחת מן המילין שמהלך הקבוע בכל יום ישאירו ט‫' | + | :<math>\scriptstyle{\color{blue}{x=10-1=9}}</math> |
| − | נמצא שבט' ימים יעמדו בשוה | + | |style="text-align:right;"|אתה תפחות אחת מן המילין שמהלך הקבוע בכל יום ישאירו ט‫' |
| + | |- | ||
| + | | | ||
| + | :We find that in 9 days they walk the same [total distance]. | ||
| + | |style="text-align:right;"|נמצא שבט' ימים יעמדו בשוה | ||
|- | |- | ||
| | | | ||
| − | :Check: | + | :<span style=color:green>'''Check:'''</span> |
| − | : | + | :The one who walks 10 miles a day walks in 9 days 90 miles. |
| + | :<math>\scriptstyle{\color{blue}{10\sdot9=90}}</math> | ||
|style="text-align:right;"|כיצד אותו שמהלך בכל יום י' מילין בט' ימים מהלך צ' מילי‫' | |style="text-align:right;"|כיצד אותו שמהלך בכל יום י' מילין בט' ימים מהלך צ' מילי‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | :For the one who walks an increasing even number of miles [each day], i.e. 2, 4, 6, 8, 10, 12, 14, 16, 18: |
| − | |style="text-align:right;"|ואותו שמהלך זוגות ר"ל ב' וד' וו' וח' וי' וי"ב וי"ד וי"ו וי"ח | + | |style="text-align:right;"|ואותו שמהלך זוגות ר"ל ב' וד' וו' וח' וי' וי"ב וי"ד וי"ו וי"ח |
| − | אמור החצי מי"ח הם ט‫' | + | |- |
| − | תרבה אותם עם י' שהוא החשבון הבא אחר ט' יהיו צ‫' | + | | |
| − | + | :Say: the half of 18 is 9 | |
| + | |style="text-align:right;"|אמור החצי מי"ח הם ט‫' | ||
| + | |- | ||
| + | | | ||
| + | :Multiply it by 10, which is the number that follows 9; it is 90. | ||
| + | |style="text-align:right;"|תרבה אותם עם י' שהוא החשבון הבא אחר ט' יהיו צ‫' | ||
|- | |- | ||
| | | | ||
| − | :(the number of miles | + | :We find that in 9 days they walk the same [total distance]. |
| − | :<math>\scriptstyle ax=\sum_{i=1}^x 2i\longrightarrow x=a-1</math> | + | |style="text-align:right;"|נמצא שבט' ימים יעמדו בשוה |
| + | |- | ||
| + | | colspan="2"| | ||
| + | :<math>\scriptstyle{\color{blue}{2+4+6+8+10+12+14+16+18=\left(18\sdot\frac{1}{2}\right)\sdot\left(9+1\right)=9\sdot10=90}}</math> | ||
| + | |- | ||
| + | |Always subtract [one] from the [number] of miles that the one who walks the same distance [each day] walks and this is the number of days, in which they walk the same [total distance]. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{ax=\sum_{i=1}^x 2i\longrightarrow x=a-1}}</math> | ||
|style="text-align:right;"|וכן לעולם תפחות מן המיל' שמהלך הקבוע וכמספרם יהיו הימים יעמדו בשוה | |style="text-align:right;"|וכן לעולם תפחות מן המיל' שמהלך הקבוע וכמספרם יהיו הימים יעמדו בשוה | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:two men|657|5kbx}}A man is walking ten miles a day. | + | *{{#annot:two men|657|5kbx}}If it is said: A man is walking ten miles a day. |
| − | :Another man is walking one mile on the first day, three miles on the second day, five miles on the third day, seven miles on the fourth day, and so on he goes on walking [in each day] an odd number of miles | + | :Another man is walking one mile on the first day, three miles on the second day, five miles on the third day, seven miles on the fourth day, and so on he goes on walking [in each day] an odd number of miles. |
:<math>\scriptstyle10X=\sum_{i=1}^x \left(2i-1\right)</math> | :<math>\scriptstyle10X=\sum_{i=1}^x \left(2i-1\right)</math> | ||
| − | |style="text-align:right;"|ואם יאמר אדם אחד הולך בכל יום י' מילי‫'<br> | + | |style="text-align:right;"|<big>ואם יאמר</big> אדם אחד הולך בכל יום י' מילי‫'<br> |
| − | ואדם אחר הולך בכל ביום אחד א' מיל וביום ב' ג' מילי' וביום ג' ה' מילי' וביום ד' ז' מילי' וכן מוסיף והולך לעולם החשבונות נפרדים{{#annotend:5kbx}} | + | ואדם אחר הולך <s>בכל</s> ביום אחד א' מיל וביום ב' ג' מילי' וביום ג' ה' מילי' וביום ד' ז' מילי' וכן מוסיף והולך לעולם החשבונות נפרדים{{#annotend:5kbx}} |
| + | |- | ||
| + | |Know that the number of miles that the one who walks the same [distance] [each day] walks each day is the same as the number of days, in which they walk the same [total distance]. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{ax=\sum_{i=1}^x \left(2i-1\right)\longrightarrow x=a}}</math> | ||
| + | |style="text-align:right;"|דע כי כמספר המילין מהלך ק הקבוע בכל יום כן מספר הימים שיעמדו בשוה | ||
|- | |- | ||
| | | | ||
| − | : | + | :I.e. in 10 days. |
| − | :<math>\scriptstyle | + | :<math>\scriptstyle{\color{blue}{x=10}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|דהיינו בי' ימים |
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{ | + | :<span style=color:green>'''Check:'''</span> |
| − | |style="text-align:right;"| | + | :Because, the one who walks 10 miles a day walks in 10 days 100 miles. |
| + | :<math>\scriptstyle{\color{blue}{10\sdot10=100}}</math> | ||
| + | |style="text-align:right;"|כי אותו שמהלך בכל יום י' מילי' ‫<ref>36r</ref>מהלך בי' ימי' ק' מילי‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | :The one who walks 1 mile on the first day, 3 miles on the second day, and so on this way, walks 19 miles on the tenth day. |
| − | + | |style="text-align:right;"|ואותו שמהלך ביום אחד א' מיל וביום שני ג' מילי' ומוסיף והולך על הדרך הזה ביום העשירי מהלך י"ט מילי‫' | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | : | + | :The greater half of 19 is 10. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והרוב מי"ט הוא י‫' |
| − | |||
| − | והרוב מי"ט הוא י | ||
| − | |||
|- | |- | ||
| | | | ||
| + | :If you multiply 10 by itself, it is 100. | ||
| + | :<math>\scriptstyle{\color{blue}{1+3+\ldots+19=\left[\left(19+1\right)\sdot\frac{1}{2}\right]^2=10^2=100}}</math> | ||
| + | |style="text-align:right;"|ואם תרבה י' בעצמם הוא ק‫' | ||
| + | |- | ||
| + | |Do this way for any number you want. | ||
|style="text-align:right;"|ועל זה הדרך תעשה לכל חשבון שתרצה | |style="text-align:right;"|ועל זה הדרך תעשה לכל חשבון שתרצה | ||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | == Word Problems - The Rule of | + | == <span style=color:green>Word Problems - The Rule of Three</span> == |
| − | + | | | |
| − | |style="text-align:right;"|ריגולא כללית והיא מבחר כל הריגולא שבעולם | + | |- |
| + | |General Rule - A Selection of all the Rules in the World | ||
| + | |style="text-align:right;"|<big>ריגולא כללית והיא מבחר כל הריגולא שבעולם</big> | ||
|- | |- | ||
| | | | ||
| − | === Pricing Problems === | + | === <span style=color:green>Pricing Problems</span> === |
| | | | ||
|- | |- | ||
| − | !Find the Price | + | !<span style=color:green>Find the Price</span> |
| | | | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:eggs|629|3fQk}}3 eggs are worth 4 pešiṭim. | + | *{{#annot:eggs|629|3fQk}}A man says: 3 eggs are worth 4 pešiṭim. |
:How many pešiṭim are 5 eggs worth at this price? | :How many pešiṭim are 5 eggs worth at this price? | ||
:<math>\scriptstyle\frac{4}{3}=\frac{X}{5}</math> | :<math>\scriptstyle\frac{4}{3}=\frac{X}{5}</math> | ||
| − | |style="text-align:right;"|אם יאמ' אדם ג' ביצות שוי ד' פשיטים<br> | + | |style="text-align:right;"|<big>אם</big> יאמ' אדם ג' ביצות שוי ד' פשיטים<br> |
ה' ביצות לזה החשבון כמה פשיטים עולי‫'{{#annotend:3fQk}} | ה' ביצות לזה החשבון כמה פשיטים עולי‫'{{#annotend:3fQk}} | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> Do this way: return the calculation back: he asks if 3 is equal to 4, how much is 5 equal to? | |
| + | :<math>\scriptstyle{\color{blue}{3:4=5:X}}</math> | ||
|style="text-align:right;"|עשה על זה הדרך שתחזיר החשבון לאחורנית הוא שואל אם ג' שוה ד' ה' כמה שוה | |style="text-align:right;"|עשה על זה הדרך שתחזיר החשבון לאחורנית הוא שואל אם ג' שוה ד' ה' כמה שוה | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{X=\frac{5\sdot4}{3}=6+\frac{2}{3}}}</math> | + | :Say: 5 times 4; divide it by 3; it is 6 and 2-thirds. |
| + | :<math>\scriptstyle{\color{blue}{X=\frac{5\sdot4}{3}=6+\frac{2}{3}}}</math> | ||
|style="text-align:right;"|ואתה אמור ה' פעמים ד' חלקם בג' יהיו ו' וב' שלישיו‫' | |style="text-align:right;"|ואתה אמור ה' פעמים ד' חלקם בג' יהיו ו' וב' שלישיו‫' | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:general|629|FApQ}}5 | + | *{{#annot:general|629|FApQ}}If he asks: if 5 is equal to 7, how much is 9 equal to? |
:<math>\scriptstyle\frac{7}{5}=\frac{X}{9}</math> | :<math>\scriptstyle\frac{7}{5}=\frac{X}{9}</math> | ||
|style="text-align:right;"|וכן אם שואל אם ה' שוה ז' ט' כמה שוי{{#annotend:FApQ}} | |style="text-align:right;"|וכן אם שואל אם ה' שוה ז' ט' כמה שוי{{#annotend:FApQ}} | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> Say: 9 times 7 is 63. Divide it by 5 and so it is. | |
| + | :<math>\scriptstyle{\color{blue}{X=\frac{9\sdot7}{5}=\frac{63}{5}}}</math> | ||
|style="text-align:right;"|אמור ט' פעמים ז' ס"ג<br> | |style="text-align:right;"|אמור ט' פעמים ז' ס"ג<br> | ||
חלקם בה' וכן יהיו | חלקם בה' וכן יהיו | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:general|629|hM80}}11 are worth 17 | + | *{{#annot:general|629|hM80}}If he says: 11 are worth 17 |
:How much are 31 worth at this price? | :How much are 31 worth at this price? | ||
:<math>\scriptstyle\frac{17}{11}=\frac{X}{31}</math> | :<math>\scriptstyle\frac{17}{11}=\frac{X}{31}</math> | ||
| Line 2,180: | Line 2,946: | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> Say: 31 times 17; divide it by 11. | |
| − | |style="text-align:right;"|אמור ל"א פעמים י"ז וחלקם בי"א וכן על זה הדרך לעולם | + | :<math>\scriptstyle{\color{blue}{X=\frac{31\sdot17}{11}}}</math> |
| + | |style="text-align:right;"|אמור ל"א פעמים י"ז וחלקם בי"א | ||
| + | |- | ||
| + | |It is always this way. | ||
| + | |style="text-align:right;"|וכן על זה הדרך לעולם | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:kor|629|lMNX}}10 korim are worth 6 dinar. | + | *{{#annot:kor|629|lMNX}}If he says: 10 korim are worth 6 dinar. |
:How much are 4 korim worth at this price? | :How much are 4 korim worth at this price? | ||
:<math>\scriptstyle\frac{6}{10}=\frac{X}{4}</math> | :<math>\scriptstyle\frac{6}{10}=\frac{X}{4}</math> | ||
| − | |style="text-align:right;"|וכן אם יאמ' י' כורי' שוי' ו' דינרי‫'<br> | + | |style="text-align:right;"|<big>וכן אם</big> יאמ' י' כורי' שוי' ו' דינרי‫'<br> |
ד' כורי' לחשבון זה כמה שוי‫'{{#annotend:lMNX}} | ד' כורי' לחשבון זה כמה שוי‫'{{#annotend:lMNX}} | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> Say: 4 times 6 is 24. Divide it by 10; the result is 2 and 4-tenths that are 2 dinar and 2-fifths of a dinar. | |
| + | :<math>\scriptstyle{\color{blue}{X=\frac{4\sdot6}{10}=\frac{24}{10}=2+\frac{4}{10}=2+\frac{2}{5}}}</math> | ||
|style="text-align:right;"|אמור ד' פעמי' ו' כ"ד<br> | |style="text-align:right;"|אמור ד' פעמי' ו' כ"ד<br> | ||
חלקם בי' יבואו ב' וד' עשיריות שהם ב' דינרי' וב' חומשי דינר | חלקם בי' יבואו ב' וד' עשיריות שהם ב' דינרי' וב' חומשי דינר | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:sheaves|629|oN2h}}3 sheaves are worth 4 dinar. | + | *{{#annot:sheaves|629|oN2h}}If it is said: 3 sheaves are worth 4 dinar. |
:How much are 10 sheaves worth at this price? | :How much are 10 sheaves worth at this price? | ||
:<math>\scriptstyle\frac{4}{3}=\frac{X}{10}</math> | :<math>\scriptstyle\frac{4}{3}=\frac{X}{10}</math> | ||
| Line 2,203: | Line 2,974: | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> Say: 10 times 4 is 40. Divide it by 3; it is 13 dinar and a third of a dinar. | |
| + | :<math>\scriptstyle{\color{blue}{X=\frac{10\sdot4}{3}=\frac{40}{3}=13+\frac{1}{3}}}</math> | ||
|style="text-align:right;"|אמור י' פעמים ד' מ‫'<br> | |style="text-align:right;"|אמור י' פעמים ד' מ‫'<br> | ||
חלקם בג' יהיו י"ג דינרי' ושליש דינר | חלקם בג' יהיו י"ג דינרי' ושליש דינר | ||
|- | |- | ||
| − | !Find the Amount | + | !<span style=color:green>Find the Amount</span> |
| | | | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:wheat|630|1vZH}}I bought 10 korim of wheat for 6 dinar. | + | *{{#annot:wheat|630|1vZH}}Say: if it is said: I bought 10 korim of wheat for 6 dinar. |
:How many korim could I buy for 4 dinar? | :How many korim could I buy for 4 dinar? | ||
:<math>\scriptstyle\frac{6}{10}=\frac{X}{4}</math> | :<math>\scriptstyle\frac{6}{10}=\frac{X}{4}</math> | ||
| Line 2,218: | Line 2,990: | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> Say: if we find 10 korim for 6 dinar, how many korim will we find for 4 dinar? | |
| + | :<math>\scriptstyle{\color{blue}{6:10=4:X}}</math> | ||
|style="text-align:right;"|אמור אם בו' דינרי' נמצא י' כורי' חטה בד' דינרי' כמה כורי' נמצא | |style="text-align:right;"|אמור אם בו' דינרי' נמצא י' כורי' חטה בד' דינרי' כמה כורי' נמצא | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{X=\frac{4\sdot10}{6}=\frac{40}{6}=6+\frac{4}{6}=6+\frac{2}{3}}}</math> | + | :Say: 4 times 10 is 40. Divide it by 6; it is 6 and 4-sixths, which is 6 and 2-thirds. |
| + | :<math>\scriptstyle{\color{blue}{X=\frac{4\sdot10}{6}=\frac{40}{6}=6+\frac{4}{6}=6+\frac{2}{3}}}</math> | ||
|style="text-align:right;"|אמור ד' פעמי' י' מ‫'<br> | |style="text-align:right;"|אמור ד' פעמי' י' מ‫'<br> | ||
חלקם בו' יהיו ו' וד' ששיות שהם ו' וב' שלישיות | חלקם בו' יהיו ו' וד' ששיות שהם ו' וב' שלישיות | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:kor|630|I3ZQ}}6 korim of wheat are worth 4 dinar. | + | *{{#annot:kor|630|I3ZQ}}If it is said: 6 korim of wheat are worth 4 dinar. |
| − | :How many | + | :How many korim could I buy for 7 dinar? |
:<math>\scriptstyle\frac{4}{6}=\frac{7}{X}</math> | :<math>\scriptstyle\frac{4}{6}=\frac{7}{X}</math> | ||
| − | |style="text-align:right;"|וכן אם יאמר שש כורי' שוי' ד' דינרי‫'<br> | + | |style="text-align:right;"|וכן אם יאמר שש כורי' שוי' ד'‫<ref>ד': MS Ithaca ו'</ref> דינרי‫'<br> |
כמה כורי אוכל לקנות בז' דינרי{{#annotend:I3ZQ}} | כמה כורי אוכל לקנות בז' דינרי{{#annotend:I3ZQ}} | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> Say: if 4 dinar are worth 6 korim, how many korim are worth 7 dinar? | |
| + | :<math>\scriptstyle{\color{blue}{4:6=7:X}}</math> | ||
|style="text-align:right;"|אמור אם ד' דינרי' שוי' ו' כורי' ז' דינרי' כמה כורי' שוי‫' | |style="text-align:right;"|אמור אם ד' דינרי' שוי' ו' כורי' ז' דינרי' כמה כורי' שוי‫' | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{X=\frac{7\sdot6}{4}=\frac{42}{4}=10+\frac{2}{4}=10+\frac{1}{2}}}</math> | + | :Say: 7 times 6 is 42. Divide it by 4; it is 10 korim and 2-quarters that are 10 korim and half a kor. |
| + | :<math>\scriptstyle{\color{blue}{X=\frac{7\sdot6}{4}=\frac{42}{4}=10+\frac{2}{4}=10+\frac{1}{2}}}</math> | ||
|style="text-align:right;"|אמור ז' פעמים ו' מ"ב<br> | |style="text-align:right;"|אמור ז' פעמים ו' מ"ב<br> | ||
| − | חלקם בד' יהיו י' כורי' וב' רביעיות שהם י' כורי' וחצי | + | חלקם בד' יהיו <s>ד' כורי</s> י' כורי' וב' רביעיות שהם <s>ו' כורי</s> י' כורי' וחצי כור |
|- | |- | ||
| | | | ||
| − | === Employment Problems - Payment Problems === | + | === <span style=color:green>Employment Problems - Payment Problems</span> === |
| − | + | | | |
| + | |- | ||
| + | |The same for the issue of employment. | ||
|style="text-align:right;"|וכן לעניין השכירות | |style="text-align:right;"|וכן לעניין השכירות | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:one worker|612|J3UH}}I hired a worker for 30 days for 10 zuzim but he worked with me 8 days. | + | *{{#annot:one worker|612|J3UH}}Example: the one who says: I hired a worker for 30 days for 10 zuzim but he worked with me 8 days. |
:How much should his payment be? | :How much should his payment be? | ||
:<math>\scriptstyle\frac{10}{30}=\frac{X}{8}</math> | :<math>\scriptstyle\frac{10}{30}=\frac{X}{8}</math> | ||
| Line 2,256: | Line 3,034: | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> Say: if for 30 days, his payment is 10 zuz, how much is his payment for 8 days? | |
| + | :<math>\scriptstyle{\color{blue}{30:10=8:X}}</math> | ||
|style="text-align:right;"|אמור אם בל' יום יהיה שכרו י' זוז בח' ימים כמה שכרו | |style="text-align:right;"|אמור אם בל' יום יהיה שכרו י' זוז בח' ימים כמה שכרו | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{X=\frac{8\sdot10}{30}=\frac{80}{30}=2+\frac{20}{30}=2+\frac{2}{3}=3-\frac{1}{3}}}</math> dinar | + | :Say: 8 times 10 is 80. Divide it by 30; the result is 2 zuzim and 20 parts of 30, which are 2 and 2-thirds, which are 3 dinar minus a third of a dinar. |
| + | :<math>\scriptstyle{\color{blue}{X=\frac{8\sdot10}{30}=\frac{80}{30}=2+\frac{20}{30}=2+\frac{2}{3}=3-\frac{1}{3}}}</math> dinar | ||
|style="text-align:right;"|אמור ח' פעמים י' הם כזה פ‫'<br> | |style="text-align:right;"|אמור ח' פעמים י' הם כזה פ‫'<br> | ||
| − | חלקם בל' יבואו ב' זוזי' וכ' חלקי' מל | + | חלקם בל' יבואו ב' זוזי' וכ' חלקי' מל' שהם ב' וב' שלישיות שהם ג' דינרי' פחות שליש דינר |
| − | שהם ב' וב' שלישיות | ||
| − | שהם ג' דינרי' | ||
|- | |- | ||
| − | + | | | |
| − | |||
| − | |||
| − | | | ||
== Calculation of Fractions == | == Calculation of Fractions == | ||
| − | + | |style="text-align:right;"|<big>חֶשְׁבּוֹן הַשְׁבָרִים</big> | |
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | *<math>\ | + | *If you divide one into two [equal] parts, each part will be a half and the two halves are one whole. |
| + | :<math>\scriptstyle{\color{blue}{1=\frac{1}{2}+\frac{1}{2}}}</math> | ||
|style="text-align:right;"|אם תחלק האחד בב' חלקים יהיה בכל חלק מהם חצי ושני החצאי' הם אחד שלם | |style="text-align:right;"|אם תחלק האחד בב' חלקים יהיה בכל חלק מהם חצי ושני החצאי' הם אחד שלם | ||
|- | |- | ||
| | | | ||
| − | *<math>\ | + | *If you divide one into three [equal] parts, each part is a third and three thirds are one whole. |
| + | :<math>\scriptstyle{\color{blue}{1=\frac{1}{3}+\frac{1}{3}+\frac{1}{3}}}</math> | ||
|style="text-align:right;"|וכן אם החלק האחד בג' חלקי' יהיה כל חלק מהם שליש ושלש השלישיות הם אחד שלם | |style="text-align:right;"|וכן אם החלק האחד בג' חלקי' יהיה כל חלק מהם שליש ושלש השלישיות הם אחד שלם | ||
|- | |- | ||
| | | | ||
| − | *<math>\ | + | *If you divide one into four [equal] parts, each part is a quarter and four quarters are one whole. |
| + | :<math>\scriptstyle{\color{blue}{1=\frac{1}{4}+\frac{1}{4}+\frac{1}{4}+\frac{1}{4}}}</math> | ||
|style="text-align:right;"|וכן אם תחלק האחד בד' חלקי' יהיה כל אחד מהם רביע וד' הרביעיי' הם אחד שלם | |style="text-align:right;"|וכן אם תחלק האחד בד' חלקי' יהיה כל אחד מהם רביע וד' הרביעיי' הם אחד שלם | ||
|- | |- | ||
| − | | | + | |This way for all. |
|style="text-align:right;"|ועל זה הדרך הם כלם | |style="text-align:right;"|ועל זה הדרך הם כלם | ||
|- | |- | ||
| | | | ||
| − | === Addition of Fractions === | + | === <span style=color:green>Addition of Fractions</span> === |
| | | | ||
|- | |- | ||
| − | |<math>\scriptstyle\frac{1}{a}+\frac{1}{b}=\frac{\left[\frac{1}{a}\sdot\left(a\sdot b\right)\right]+\left[\frac{1}{b}\sdot\left(a\sdot b\right)\right]}{a\sdot b}</math> | + | |<math>\scriptstyle{\color{OliveGreen}{\frac{1}{a}+\frac{1}{b}=\frac{\left[\frac{1}{a}\sdot\left(a\sdot b\right)\right]+\left[\frac{1}{b}\sdot\left(a\sdot b\right)\right]}{a\sdot b}}}</math> |
| | | | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:½+⅓|677|Prkw}}<math>\scriptstyle\frac{1}{2}+\frac{1}{3}</math> | + | *{{#annot:½+⅓|677|Prkw}}If it is said: whoever has a half and a third of one thing, how much does he have of that thing? |
| + | :<math>\scriptstyle\frac{1}{2}+\frac{1}{3}</math> | ||
|style="text-align:right;"|ואם יאמ' מי שיש לו החצי והשליש מדבר אחד כמה חלק יש לו מן הדבר ההוא{{#annotend:Prkw}} | |style="text-align:right;"|ואם יאמ' מי שיש לו החצי והשליש מדבר אחד כמה חלק יש לו מן הדבר ההוא{{#annotend:Prkw}} | ||
|- | |- | ||
| | | | ||
| − | :<span style=color: | + | :<span style=color:green>'''Common denominator:'''</span> Say: a half and a third are found in 2 times 3, which is 6. |
| − | |style="text-align:right;"|אמור חצי ושליש ימצא בב' פעמים ג' שהם ו‫' | + | :<math>\scriptstyle{\color{blue}{2\sdot3=6}}</math> |
| + | |style="text-align:right;"|אמור חצי ושליש ‫<ref>36v</ref>ימצא בב' פעמים ג' שהם ו‫' | ||
|- | |- | ||
| | | | ||
| + | :Its half is 3 and its third is 2. If you sum them, they are 5. So, the one who has a half and a third of the thing, has 5 parts of 6 of that thing. | ||
:<math>\scriptstyle{\color{blue}{\frac{1}{2}+\frac{1}{3}=\frac{\left(\frac{1}{2}\sdot6\right)+\left(\frac{1}{3}\sdot6\right)}{6}=\frac{3+2}{6}=\frac{5}{6}}}</math> | :<math>\scriptstyle{\color{blue}{\frac{1}{2}+\frac{1}{3}=\frac{\left(\frac{1}{2}\sdot6\right)+\left(\frac{1}{3}\sdot6\right)}{6}=\frac{3+2}{6}=\frac{5}{6}}}</math> | ||
|style="text-align:right;"|חציי ג' ושלישיתו ב' ואם תקבצו יהיו ה' הרי שאותו שיש לו החצי והשליש מן הדבר יש לו ה' חלקי' מו' מן הדבר ההוא | |style="text-align:right;"|חציי ג' ושלישיתו ב' ואם תקבצו יהיו ה' הרי שאותו שיש לו החצי והשליש מן הדבר יש לו ה' חלקי' מו' מן הדבר ההוא | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:⅓+¼|677|595p}}<math>\scriptstyle\frac{1}{3}+\frac{1}{4}</math> | + | *{{#annot:⅓+¼|677|595p}}Whoever has a third and a quarter of the thing, how much does he have of that thing? |
| − | |style="text-align:right;"|ואם מי שיש לו השליש והרביעי מן הדבר כמה חלק יש לו ממנו{{#annotend:595p}} | + | :<math>\scriptstyle\frac{1}{3}+\frac{1}{4}</math> |
| + | |style="text-align:right;"|<big>ואם</big> מי שיש לו השליש והרביעי מן הדבר כמה חלק יש לו ממנו{{#annotend:595p}} | ||
|- | |- | ||
| | | | ||
| − | :<span style=color: | + | :<span style=color:green>'''Common denominator:'''</span> Say: a third and a quarter are found in 3 times 4, which is 12. |
| + | :<math>\scriptstyle{\color{blue}{3\sdot4=12}}</math> | ||
|style="text-align:right;"|אמור שליש ורביע ימצא בג' פעמים ד' שהם י"ב | |style="text-align:right;"|אמור שליש ורביע ימצא בג' פעמים ד' שהם י"ב | ||
|- | |- | ||
| | | | ||
| + | :Its third is 4 and its quarter is 3. If you sum them, they are 7. So, the one who has a third and a quarter of the thing, has 7 parts of 12 of that thing. | ||
:<math>\scriptstyle{\color{blue}{\frac{1}{3}+\frac{1}{4}=\frac{\left(\frac{1}{3}\sdot12\right)+\left(\frac{1}{4}\sdot12\right)}{12}=\frac{4+3}{12}=\frac{7}{12}}}</math> | :<math>\scriptstyle{\color{blue}{\frac{1}{3}+\frac{1}{4}=\frac{\left(\frac{1}{3}\sdot12\right)+\left(\frac{1}{4}\sdot12\right)}{12}=\frac{4+3}{12}=\frac{7}{12}}}</math> | ||
|style="text-align:right;"|השליש מהם הם ד' והרביעי הוא ג' ואם תקבצם הם ז' הרי שמי שיש לו השליש והרביע מן הדבר יש לו ז' חלקי' מי"ב מן הדבר ההוא | |style="text-align:right;"|השליש מהם הם ד' והרביעי הוא ג' ואם תקבצם הם ז' הרי שמי שיש לו השליש והרביע מן הדבר יש לו ז' חלקי' מי"ב מן הדבר ההוא | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:⅓+⅕|677|Asm1}}<math>\scriptstyle\frac{1}{3}+\frac{1}{5}</math> | + | *{{#annot:⅓+⅕|677|Asm1}}Whoever has a third and a fifth of the thing. |
| + | :<math>\scriptstyle\frac{1}{3}+\frac{1}{5}</math> | ||
|style="text-align:right;"|וכן מי שיש לו השליש והחומש מן הדבר{{#annotend:Asm1}} | |style="text-align:right;"|וכן מי שיש לו השליש והחומש מן הדבר{{#annotend:Asm1}} | ||
|- | |- | ||
| | | | ||
| − | :<span style=color: | + | :<span style=color:green>'''Common denominator:'''</span> Say: a third and a fifth are found in 3 times 5, which is 15. |
| + | :<math>\scriptstyle{\color{blue}{3\sdot5=15}}</math> | ||
|style="text-align:right;"|אמור שליש וחומש ימצא בג' פעמים ה' שהם ט"ו | |style="text-align:right;"|אמור שליש וחומש ימצא בג' פעמים ה' שהם ט"ו | ||
|- | |- | ||
| | | | ||
| + | :Its third is 5 and its fifth is 3. Together they are 8. So, the one who has a third and a fifth of the thing, has 8 parts of 15 of that thing. | ||
:<math>\scriptstyle{\color{blue}{\frac{1}{3}+\frac{1}{5}=\frac{\left(\frac{1}{3}\sdot15\right)+\left(\frac{1}{5}\sdot15\right)}{15}=\frac{5+3}{15}=\frac{8}{15}}}</math> | :<math>\scriptstyle{\color{blue}{\frac{1}{3}+\frac{1}{5}=\frac{\left(\frac{1}{3}\sdot15\right)+\left(\frac{1}{5}\sdot15\right)}{15}=\frac{5+3}{15}=\frac{8}{15}}}</math> | ||
|style="text-align:right;"|השליש מהם הוא ה' והחומש ג' ושניהם יחד הם ח' אם כן מי שיש לו השליש והחומש מן הדבר יש לו ח' חלקים מט"ו מן הדבר ההוא | |style="text-align:right;"|השליש מהם הוא ה' והחומש ג' ושניהם יחד הם ח' אם כן מי שיש לו השליש והחומש מן הדבר יש לו ח' חלקים מט"ו מן הדבר ההוא | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:¼+⅕|677|nQTO}}<math>\scriptstyle\frac{1}{4}+\frac{1}{5}</math> | + | *{{#annot:¼+⅕|677|nQTO}}Whoever has a quarter and a fifth of the thing. |
| + | :<math>\scriptstyle\frac{1}{4}+\frac{1}{5}</math> | ||
|style="text-align:right;"|וכן מי שיש לו הרביע והחומש מן הדבר{{#annotend:nQTO}} | |style="text-align:right;"|וכן מי שיש לו הרביע והחומש מן הדבר{{#annotend:nQTO}} | ||
|- | |- | ||
| | | | ||
| − | :<span style=color: | + | :<span style=color:green>'''Common denominator:'''</span> Say: a quarter and a fifth are found in 4 times 5, which is 20. |
| + | :<math>\scriptstyle{\color{blue}{4\sdot5=20}}</math> | ||
|style="text-align:right;"|אמור רביע וחומש ימצא בד' פעמי' ה' שהם כ‫' | |style="text-align:right;"|אמור רביע וחומש ימצא בד' פעמי' ה' שהם כ‫' | ||
|- | |- | ||
| | | | ||
| + | :Its quarter is 5 and its fifth is 4. Together they are 9. So, he has 9 parts of 20 of that thing. | ||
:<math>\scriptstyle{\color{blue}{\frac{1}{4}+\frac{1}{5}=\frac{\left(\frac{1}{4}\sdot20\right)+\left(\frac{1}{5}\sdot20\right)}{20}=\frac{5+4}{20}=\frac{9}{20}}}</math> | :<math>\scriptstyle{\color{blue}{\frac{1}{4}+\frac{1}{5}=\frac{\left(\frac{1}{4}\sdot20\right)+\left(\frac{1}{5}\sdot20\right)}{20}=\frac{5+4}{20}=\frac{9}{20}}}</math> | ||
| − | |style="text-align:right;"|הרביע הוא ה' והחומש הוא ד' ושניהם יחד הם ט' אם כן יש לו ט' חלקים מכ' מן הדבר ההוא | + | |style="text-align:right;"|הרביע הוא‫<ref>הוא: MS Ithaca הם</ref> ה' והחומש הוא ד'‫<ref>והחומש הוא ד': MS Ithaca om.</ref> ושניהם יחד הם ט'‫<ref>ט': MS Ithaca ד'</ref> אם כן יש לו ט' חלקים מכ' מן הדבר ההוא |
|- | |- | ||
| | | | ||
| − | *{{#annot:⅓+⅐|677|c6OW}}<math>\scriptstyle\frac{1}{3}+\frac{1}{7}</math> | + | *{{#annot:⅓+⅐|677|c6OW}}Whoever has a third and a seventh of the thing. |
| + | :<math>\scriptstyle\frac{1}{3}+\frac{1}{7}</math> | ||
|style="text-align:right;"|וכן מי שיש לו שליש ושביע מן הדבר{{#annotend:c6OW}} | |style="text-align:right;"|וכן מי שיש לו שליש ושביע מן הדבר{{#annotend:c6OW}} | ||
|- | |- | ||
| | | | ||
| − | :<span style=color: | + | :<span style=color:green>'''Common denominator:'''</span> Say: a third and a seventh are found in 3 times 7, which is 21. |
| + | :<math>\scriptstyle{\color{blue}{3\sdot7=21}}</math> | ||
|style="text-align:right;"|אמור שליש ושביע ימצאו בג' פעמים ז' שהם כ"א | |style="text-align:right;"|אמור שליש ושביע ימצאו בג' פעמים ז' שהם כ"א | ||
|- | |- | ||
| | | | ||
| + | :Its third is 7 and its seventh is 3. Together they are 10. So, he has 10 parts of 21. | ||
:<math>\scriptstyle{\color{blue}{\frac{1}{3}+\frac{1}{7}=\frac{\left(\frac{1}{3}\sdot21\right)+\left(\frac{1}{7}\sdot21\right)}{21}=\frac{7+3}{21}=\frac{10}{21}}}</math> | :<math>\scriptstyle{\color{blue}{\frac{1}{3}+\frac{1}{7}=\frac{\left(\frac{1}{3}\sdot21\right)+\left(\frac{1}{7}\sdot21\right)}{21}=\frac{7+3}{21}=\frac{10}{21}}}</math> | ||
| − | |style="text-align:right;"|השליש ז' והשביעי ג' ושניהם יחד הם י' א"כ יש לו י' חלקי' מכ"א | + | |style="text-align:right;"|השליש ז' והשביעי ג' ושניהם יחד הם י' א"כ יש לו י'‫<ref>י': MS Ithaca ו'</ref> חלקי' מכ"א |
|- | |- | ||
| | | | ||
| − | *{{#annot:⅓+¼+⅕+⅙|677|pvs7}}<math>\scriptstyle\frac{1}{3}+\frac{1}{4}+\frac{1}{5}+\frac{1}{6}</math> | + | *{{#annot:⅓+¼+⅕+⅙|677|pvs7}}Whoever has a third, a quarter, a fifth, and a sixth of the thing. |
| + | :<math>\scriptstyle\frac{1}{3}+\frac{1}{4}+\frac{1}{5}+\frac{1}{6}</math> | ||
|style="text-align:right;"|וכן מי שיש לו שליש ורביע וחומש ושתות מן הדבר{{#annotend:pvs7}} | |style="text-align:right;"|וכן מי שיש לו שליש ורביע וחומש ושתות מן הדבר{{#annotend:pvs7}} | ||
|- | |- | ||
| | | | ||
| − | :<span style=color: | + | :<span style=color:green>'''Common denominator:'''</span> Say: a third, a quarter, a fifth and a sixth are found in 60. |
|style="text-align:right;"|אמור שליש ורביע וחומש ושתות אם ימצאו בס‫' | |style="text-align:right;"|אמור שליש ורביע וחומש ושתות אם ימצאו בס‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | :Its third is 20, its quarter is 15, its fifth is 12 and its sixth is 10. If you sum them, they are 57. So, he has 57 parts of 60 of that thing. |
|style="text-align:right;"|השליש הוא כ' הרבי' ט"ו והחומש י"ב והשתות י' ואם תקבצם יהיו נ"ז הרי שיש לו נ"ז חלקים מס' מן הדבר ההוא | |style="text-align:right;"|השליש הוא כ' הרבי' ט"ו והחומש י"ב והשתות י' ואם תקבצם יהיו נ"ז הרי שיש לו נ"ז חלקים מס' מן הדבר ההוא | ||
| − | |} | + | |- |
| − | { | + | | colspan="2"| |
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{3}+\frac{1}{4}+\frac{1}{5}+\frac{1}{6}=\frac{\left(\frac{1}{3}\sdot60\right)+\left(\frac{1}{4}\sdot60\right)+\left(\frac{1}{5}\sdot60\right)+\left(\frac{1}{6}\sdot60\right)}{60}=\frac{20+15+12+10}{60}=\frac{57}{60}}}</math> | ||
|- | |- | ||
| | | | ||
| − | === Subtraction of Fractions === | + | === <span style=color:green>Subtraction of Fractions</span> === |
| | | | ||
|- | |- | ||
| − | |<math>\scriptstyle\frac{1}{a}-\frac{1}{b}=\frac{\left[\frac{1}{a}\sdot\left(a\sdot b\right)\right]-\left[\frac{1}{b}\sdot\left(a\sdot b\right)\right]}{a\sdot b}</math> | + | |<math>\scriptstyle{\color{OliveGreen}{\frac{1}{a}-\frac{1}{b}=\frac{\left[\frac{1}{a}\sdot\left(a\sdot b\right)\right]-\left[\frac{1}{b}\sdot\left(a\sdot b\right)\right]}{a\sdot b}}}</math> |
| | | | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:⅓-¼|678|Gem8}}<math>\scriptstyle\frac{1}{3}-\frac{1}{4}</math> | + | *{{#annot:⅓-¼|678|Gem8}}If a man says: by how much is the third greater than the quarter? |
| + | :<math>\scriptstyle\frac{1}{3}-\frac{1}{4}</math> | ||
|style="text-align:right;"|אמנם אם יאמר אדם כמה הוא יותר השליש מן הרביע{{#annotend:Gem8}} | |style="text-align:right;"|אמנם אם יאמר אדם כמה הוא יותר השליש מן הרביע{{#annotend:Gem8}} | ||
|- | |- | ||
| | | | ||
| − | :<span style=color: | + | :<span style=color:green>'''Common denominator:'''</span> Do this way, say: a third and a quarter are found in 3 times 4, which is 12. |
| + | :<math>\scriptstyle{\color{blue}{3\sdot4=12}}</math> | ||
|style="text-align:right;"|עשה על זה הדרך אמור שליש ורביע ימצאו בג' פעמי' ד' שהם י"ב | |style="text-align:right;"|עשה על זה הדרך אמור שליש ורביע ימצאו בג' פעמי' ד' שהם י"ב | ||
|- | |- | ||
| | | | ||
| + | :Its third is 4 and its quarter is 3. By how much is 4, which is the third, greater than 3, which is the quarter? By 1. So, the third is greater than the quarter by 1 part of 12. | ||
:<math>\scriptstyle{\color{blue}{\frac{1}{3}-\frac{1}{4}=\frac{\left(\frac{1}{3}\sdot12\right)-\left(\frac{1}{4}\sdot12\right)}{12}=\frac{4-3}{12}=\frac{1}{12}}}</math> | :<math>\scriptstyle{\color{blue}{\frac{1}{3}-\frac{1}{4}=\frac{\left(\frac{1}{3}\sdot12\right)-\left(\frac{1}{4}\sdot12\right)}{12}=\frac{4-3}{12}=\frac{1}{12}}}</math> | ||
|style="text-align:right;"|השליש הוא ד' והרביעי הוא ג' כמה יותר ד' שהוא השליש מג' שהוא הרביעי א' א"כ השליש הוא יותר מן הרביע א' חלק מי"ב | |style="text-align:right;"|השליש הוא ד' והרביעי הוא ג' כמה יותר ד' שהוא השליש מג' שהוא הרביעי א' א"כ השליש הוא יותר מן הרביע א' חלק מי"ב | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:¼-⅕|678|wkXD}}<math>\scriptstyle\frac{1}{4}-\frac{1}{5}</math> | + | *{{#annot:¼-⅕|678|wkXD}} If a man says: by how much is the quarter greater than the fifth? |
| + | :<math>\scriptstyle\frac{1}{4}-\frac{1}{5}</math> | ||
|style="text-align:right;"|וכן אם יאמר אדם כמה הוא יותר הרביע מן החומש{{#annotend:wkXD}} | |style="text-align:right;"|וכן אם יאמר אדם כמה הוא יותר הרביע מן החומש{{#annotend:wkXD}} | ||
|- | |- | ||
| | | | ||
| − | :<span style=color: | + | :<span style=color:green>'''Common denominator:'''</span> Say: a quarter and a fifth are found in 4 times 5, which is 20. |
| + | :<math>\scriptstyle{\color{blue}{4\sdot5=20}}</math> | ||
|style="text-align:right;"|אמור רביע וחומש ימצאו בד' פעמי' ה' שהוא כ‫' | |style="text-align:right;"|אמור רביע וחומש ימצאו בד' פעמי' ה' שהוא כ‫' | ||
|- | |- | ||
| | | | ||
| + | :Its quarter is 5 and its fifth is 4. By how much is 5 greater than 4? By 1. So, the quarter is greater than the fifth by 1 part of twenty. | ||
:<math>\scriptstyle{\color{blue}{\frac{1}{4}-\frac{1}{5}=\frac{\left(\frac{1}{4}\sdot20\right)-\left(\frac{1}{5}\sdot20\right)}{20}=\frac{5-4}{20}=\frac{1}{20}}}</math> | :<math>\scriptstyle{\color{blue}{\frac{1}{4}-\frac{1}{5}=\frac{\left(\frac{1}{4}\sdot20\right)-\left(\frac{1}{5}\sdot20\right)}{20}=\frac{5-4}{20}=\frac{1}{20}}}</math> | ||
|style="text-align:right;"|הרביעי הוא ה' והחומש הוא ד' כמה הוא יותר ה' מד' א' אם כן הרביע הוא יותר מן החומש א' חלק מעשרים | |style="text-align:right;"|הרביעי הוא ה' והחומש הוא ד' כמה הוא יותר ה' מד' א' אם כן הרביע הוא יותר מן החומש א' חלק מעשרים | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:⅓-⅐|678|ebvM}}<math>\scriptstyle\frac{1}{3}-\frac{1}{7}</math> | + | *{{#annot:⅓-⅐|678|ebvM}} If it is said: by how much is the third greater than the seventh? |
| + | :<math>\scriptstyle\frac{1}{3}-\frac{1}{7}</math> | ||
|style="text-align:right;"|וכן אם יאמר כמה הוא יותר השליש מן השביע{{#annotend:ebvM}} | |style="text-align:right;"|וכן אם יאמר כמה הוא יותר השליש מן השביע{{#annotend:ebvM}} | ||
|- | |- | ||
| | | | ||
| − | :<span style=color: | + | :<span style=color:green>'''Common denominator:'''</span> Say: a third and a seventh are found in 3 times 7, which is 21. |
| + | :<math>\scriptstyle{\color{blue}{3\sdot7=21}}</math> | ||
|style="text-align:right;"|אמור שליש ושביע ימצאו בג' פעמים ז' שהם כ"א | |style="text-align:right;"|אמור שליש ושביע ימצאו בג' פעמים ז' שהם כ"א | ||
|- | |- | ||
| | | | ||
| + | :Its third is 7 and its seventh is 3. By how much is 7 greater than 3? By 4. So, the third is greater than the seventh by 4 parts of 21. | ||
:<math>\scriptstyle{\color{blue}{\frac{1}{3}-\frac{1}{7}=\frac{\left(\frac{1}{3}\sdot21\right)-\left(\frac{1}{7}\sdot21\right)}{21}=\frac{7-3}{21}=\frac{4}{21}}}</math> | :<math>\scriptstyle{\color{blue}{\frac{1}{3}-\frac{1}{7}=\frac{\left(\frac{1}{3}\sdot21\right)-\left(\frac{1}{7}\sdot21\right)}{21}=\frac{7-3}{21}=\frac{4}{21}}}</math> | ||
|style="text-align:right;"|השליש הוא ז' השביע הוא ג' כמה הוא יותר ז' על ג' ד' וא"כ השליש יותר מן השביע ד' חלקי' מכ"א | |style="text-align:right;"|השליש הוא ז' השביע הוא ג' כמה הוא יותר ז' על ג' ד' וא"כ השליש יותר מן השביע ד' חלקי' מכ"א | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:(⅓+¼)-(⅕+⅙)|678|e3Ts}}<math>\scriptstyle\left(\frac{1}{3}+\frac{1}{4}\right)-\left(\frac{1}{5}+\frac{1}{6}\right)</math> | + | *{{#annot:(⅓+¼)-(⅕+⅙)|678|e3Ts}} If it is said: by how much are the third and the quarter greater than the fifth and the eighth? |
| + | :<math>\scriptstyle\left(\frac{1}{3}+\frac{1}{4}\right)-\left(\frac{1}{5}+\frac{1}{6}\right)</math> | ||
|style="text-align:right;"|ואם יאמר כמה הם יותר השליש והרביע מן החומש ושתות{{#annotend:e3Ts}} | |style="text-align:right;"|ואם יאמר כמה הם יותר השליש והרביע מן החומש ושתות{{#annotend:e3Ts}} | ||
|- | |- | ||
| | | | ||
| − | :<span style=color: | + | :<span style=color:green>'''Common denominator:'''</span> Say: a third, a quarter, a fifth and a sixth are found in 60. |
|style="text-align:right;"|אמור שליש ורביע וחומש ושתות ימצאו בס‫' | |style="text-align:right;"|אמור שליש ורביע וחומש ושתות ימצאו בס‫' | ||
|- | |- | ||
| | | | ||
| + | :Its third is 20 and its quarter is 15. If you sum them, they are 35. The fifth of 60 is 12 and its sixth is 10. If you sum them, they are 22. By how much is 35, which is the third and the quarter, greater than 22, which is the fifth and the sixth? By 13. So, the third and the quarter are greater than the fifth and the sixth by 13 parts of 60. | ||
| + | |style="text-align:right;"|השליש הוא כ' הרביע ט"ו אם תקבצם יהיו ל"ה והחומש מס' הוא ל"ב י"ב השתות י' אם תקבצם יהיו כ"ב כמה הם יותר ל"ה שהם השליש והרביע מכ"ב שהם החומש והשתות י"ג אם כן השליש והרביע הם יותר מן החומש והשתות י"ג חלקים מס‫' | ||
| + | |- | ||
| + | | colspan="2"| | ||
:<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle\left(\frac{1}{3}+\frac{1}{4}\right)-\left(\frac{1}{5}+\frac{1}{6}\right)&\scriptstyle=\frac{\left[\left(\frac{1}{3}\sdot60\right)+\left(\frac{1}{4}\sdot60\right)\right]-\left[\left(\frac{1}{5}\sdot60\right)+\left(\frac{1}{6}\sdot60\right)\right]}{60}\\&\scriptstyle=\frac{\left(20+15\right)-\left(12+10\right)}{60}=\frac{35-22}{60}=\frac{13}{60}\\\end{align}}}</math> | :<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle\left(\frac{1}{3}+\frac{1}{4}\right)-\left(\frac{1}{5}+\frac{1}{6}\right)&\scriptstyle=\frac{\left[\left(\frac{1}{3}\sdot60\right)+\left(\frac{1}{4}\sdot60\right)\right]-\left[\left(\frac{1}{5}\sdot60\right)+\left(\frac{1}{6}\sdot60\right)\right]}{60}\\&\scriptstyle=\frac{\left(20+15\right)-\left(12+10\right)}{60}=\frac{35-22}{60}=\frac{13}{60}\\\end{align}}}</math> | ||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | === Fractions of Fractions === | + | === <span style=color:green>Fractions of Fractions</span> === |
| | | | ||
|- | |- | ||
| − | |<math>\scriptstyle\frac{1}{a}\sdot\frac{1}{b}=\frac{\frac{1}{a}\sdot\left[\frac{1}{b}\sdot\left(a\sdot b\right)\right]}{a\sdot b}</math> | + | |<math>\scriptstyle{\color{OliveGreen}{\frac{1}{a}\sdot\frac{1}{b}=\frac{\frac{1}{a}\sdot\left[\frac{1}{b}\sdot\left(a\sdot b\right)\right]}{a\sdot b}}}</math> |
| | | | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:⅓·¼|668|KdCj}}<math>\scriptstyle\frac{1}{3}\sdot\frac{1}{4}</math> | + | *{{#annot:⅓·¼|668|KdCj}}If it is said: whoever has a third of a quarter of the thing, how much does he have of [that thing]? |
| + | :<math>\scriptstyle\frac{1}{3}\sdot\frac{1}{4}</math> | ||
|style="text-align:right;"|אמנם אם יאמר מי שיש לו השליש מן הרביע מן הדבר כמה חלק יש לו ממנו{{#annotend:KdCj}} | |style="text-align:right;"|אמנם אם יאמר מי שיש לו השליש מן הרביע מן הדבר כמה חלק יש לו ממנו{{#annotend:KdCj}} | ||
|- | |- | ||
| | | | ||
| − | :<span style=color: | + | :<span style=color:green>'''Common denominator:'''</span> Say: a third and a quarter are found in 12. |
|style="text-align:right;"|אמור שליש ורביע ימצא בי"ב | |style="text-align:right;"|אמור שליש ורביע ימצא בי"ב | ||
|- | |- | ||
| | | | ||
| + | :Whoever has a quarter of 12, has 3 parts of 12. Whoever has a third of these three that are a quarter, has 1 part of 12. | ||
:<math>\scriptstyle{\color{blue}{\frac{1}{3}\sdot\frac{1}{4}=\frac{\frac{1}{3}\sdot\left(\frac{1}{4}\sdot12\right)}{12}=\frac{\frac{1}{3}\sdot3}{12}=\frac{1}{12}}}</math> | :<math>\scriptstyle{\color{blue}{\frac{1}{3}\sdot\frac{1}{4}=\frac{\frac{1}{3}\sdot\left(\frac{1}{4}\sdot12\right)}{12}=\frac{\frac{1}{3}\sdot3}{12}=\frac{1}{12}}}</math> | ||
|style="text-align:right;"|ומי שיש לו הרביע מי"ב יש לו ג' חלקים מי"ב ומי שיש לו השליש מאלו הג' שהם הרביע יהיה לו א' חלק מי"ב | |style="text-align:right;"|ומי שיש לו הרביע מי"ב יש לו ג' חלקים מי"ב ומי שיש לו השליש מאלו הג' שהם הרביע יהיה לו א' חלק מי"ב | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:⅓·⅕|668|dqBo}}<math>\scriptstyle\frac{1}{3}\sdot\frac{1}{5}</math> | + | *{{#annot:⅓·⅕|668|dqBo}}If it is said: whoever has a third of a fifth [of the thing], how much does he have of [that thing]? |
| + | :<math>\scriptstyle\frac{1}{3}\sdot\frac{1}{5}</math> | ||
|style="text-align:right;"|ואם יאמר מי שיש לו השליש מן החומש כמה חלק יש לו{{#annotend:dqBo}} | |style="text-align:right;"|ואם יאמר מי שיש לו השליש מן החומש כמה חלק יש לו{{#annotend:dqBo}} | ||
|- | |- | ||
| | | | ||
| − | :<span style=color: | + | :<span style=color:green>'''Common denominator:'''</span> Say: a third and a fifth are found in 3 times 5, which is 15. |
| + | :<math>\scriptstyle{\color{blue}{3\sdot5=15}}</math> | ||
|style="text-align:right;"|אמור שליש וחומש ימצא בג' פעמים ה' שהם ט"ו | |style="text-align:right;"|אמור שליש וחומש ימצא בג' פעמים ה' שהם ט"ו | ||
|- | |- | ||
| | | | ||
| + | :The fifth of 15 is 3. Whoever has a third of the 3, which is the fifth, has 1 part of 15. | ||
:<math>\scriptstyle{\color{blue}{\frac{1}{3}\sdot\frac{1}{5}=\frac{\frac{1}{3}\sdot\left(\frac{1}{5}\sdot15\right)}{15}=\frac{\frac{1}{3}\sdot3}{15}=\frac{1}{15}}}</math> | :<math>\scriptstyle{\color{blue}{\frac{1}{3}\sdot\frac{1}{5}=\frac{\frac{1}{3}\sdot\left(\frac{1}{5}\sdot15\right)}{15}=\frac{\frac{1}{3}\sdot3}{15}=\frac{1}{15}}}</math> | ||
|style="text-align:right;"|החומש מט"ו הם ג' ומי שיש לו השליש מג' שהוא החומש יש לו א' חלק מט"ו | |style="text-align:right;"|החומש מט"ו הם ג' ומי שיש לו השליש מג' שהוא החומש יש לו א' חלק מט"ו | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:⅕·⅑|668|3ST2}}<math>\scriptstyle\frac{1}{5}\sdot\frac{1}{9}</math> | + | *{{#annot:⅕·⅑|668|3ST2}}Whoever has a fifth of a ninth. |
| − | |style="text-align:right;"|וכן מי שיש לו החומש מן התשיעית{{#annotend:3ST2}} | + | :<math>\scriptstyle\frac{1}{5}\sdot\frac{1}{9}</math> |
| + | |style="text-align:right;"|‫<ref>37r</ref>וכן מי שיש לו החומש מן התשיעית{{#annotend:3ST2}} | ||
|- | |- | ||
| | | | ||
| − | :<span style=color: | + | :<span style=color:green>'''Common denominator:'''</span> Say: a fifth and a ninth are found in 5 times 9, which is 45. |
| + | :<math>\scriptstyle{\color{blue}{5\sdot9=45}}</math> | ||
|style="text-align:right;"|אמור חומש ותשיע ימצא בה' פעמים ט' שהם מ"ה | |style="text-align:right;"|אמור חומש ותשיע ימצא בה' פעמים ט' שהם מ"ה | ||
|- | |- | ||
| | | | ||
| + | :The ninth of 45 is 5 and the fifth of 5 is 1. So, whoever has a fifth of a ninth, has 1 part of 45 of that thing. | ||
:<math>\scriptstyle{\color{blue}{\frac{1}{5}\sdot\frac{1}{9}=\frac{\frac{1}{5}\sdot\left(\frac{1}{9}\sdot45\right)}{45}=\frac{\frac{1}{5}\sdot5}{45}=\frac{1}{45}}}</math> | :<math>\scriptstyle{\color{blue}{\frac{1}{5}\sdot\frac{1}{9}=\frac{\frac{1}{5}\sdot\left(\frac{1}{9}\sdot45\right)}{45}=\frac{\frac{1}{5}\sdot5}{45}=\frac{1}{45}}}</math> | ||
|style="text-align:right;"|והתשיעי ממ"ה הוא ה' והחומש מה' הוא א' א"כ מי שיש לו החומש מן התשיעית יש לו א' חלק ממ"ה מן הדבר ההוא | |style="text-align:right;"|והתשיעי ממ"ה הוא ה' והחומש מה' הוא א' א"כ מי שיש לו החומש מן התשיעית יש לו א' חלק ממ"ה מן הדבר ההוא | ||
|- | |- | ||
| | | | ||
| − | === Conversion of fractions === | + | |
| + | === <span style=color:green>Conversion of fractions</span> === | ||
| | | | ||
|- | |- | ||
| | | | ||
| − | *How many tenths there are in three eighths | + | *If a man says: How many tenths there are in three eighths? |
|style="text-align:right;"|אם יאמר אדם ג' שמיניות כמה עשיריות הם | |style="text-align:right;"|אם יאמר אדם ג' שמיניות כמה עשיריות הם | ||
|- | |- | ||
| | | | ||
| − | :<span style=color: | + | :Do this way: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|עשה על זה הדרך |
| + | |- | ||
| + | | | ||
| + | :<span style=color:green>'''Common denominator:'''</span> Say: an eighth and a tenth are found in 8 times 10, which is 80. | ||
| + | :<math>\scriptstyle{\color{blue}{8\sdot10=80}}</math> | ||
| + | |style="text-align:right;"|אמור שמינית ועשירי' ימצאו בח' פעמי' י' שהם פ‫' | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\frac{3}{8}\sdot80=30}}</math> | + | :3-eighths are 30. |
| + | :<math>\scriptstyle{\color{blue}{\frac{3}{8}\sdot80=30}}</math> | ||
|style="text-align:right;"|וג' שמיניות הם ל‫' | |style="text-align:right;"|וג' שמיניות הם ל‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | :Because 10 is one-eighth of 80. |
| − | |style="text-align:right;"|כי י' הוא שמינית פ‫' | + | :<math>\scriptstyle{\color{blue}{\frac{1}{8}\sdot80=10}}</math> |
| + | |style="text-align:right;"|כי י' הוא שמינית פ'‫<ref>וג'...שמינית פ': MS Ithaca om.</ref> | ||
|- | |- | ||
| | | | ||
| − | : | + | :And 8 is one-tenth of 80. |
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{10}\sdot80=8}}</math> | ||
|style="text-align:right;"|וח' הוא עשירית פ‫' | |style="text-align:right;"|וח' הוא עשירית פ‫' | ||
|- | |- | ||
| | | | ||
| + | :If you wish to know how many tenths are the 3-eighth of 80, divide 30, which is 3-eighths, by 8, which is one-tenth of 80; it is 3-tenths and 6 parts of 80. | ||
:<math>\scriptstyle{\color{blue}{\frac{3}{8}=\frac{\frac{3}{8}\sdot80}{80}=\frac{30}{80}=\frac{\frac{30}{8}}{10}=\frac{3}{10}+\frac{6}{80}}}</math> | :<math>\scriptstyle{\color{blue}{\frac{3}{8}=\frac{\frac{3}{8}\sdot80}{80}=\frac{30}{80}=\frac{\frac{30}{8}}{10}=\frac{3}{10}+\frac{6}{80}}}</math> | ||
| − | |style="text-align:right;"|ואם תרצה לידע כמה עשיריות הם הג' שמיניות מפ' חלק הל' שהוא הג' שמיניות חלקם בח' שהוא עשירית פ' הם ג' עשריות וו' ואותם הו' הם ו' חלקים מפ‫' | + | |style="text-align:right;"|ואם תרצה לידע כמה עשיריות הם הג' שמיניות מפ' חלק הל' שהוא הג' שמיניות חלקם‫<ref>שהוא הג' שמיניות חלקם: MS Ithaca om.</ref> בח' שהוא עשירית פ' הם ג' עשריות וו' ואותם הו' הם ו' חלקים מפ'‫<ref>שהוא עשירית פ'...חלקים מפ': MS Ithaca יהיה ג‫'</ref> |
|- | |- | ||
| | | | ||
| − | === Operations with fractions - numerator greater than 1 === | + | === <span style=color:green>Operations with fractions - numerator greater than 1</span> === |
| | | | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:⅜+⁷/₁₀|677|tIym}}<math>\scriptstyle\frac{3}{8}+\frac{7}{10}</math> | + | *{{#annot:⅜+⁷/₁₀|677|tIym}}If one says: add 3-eighths to 7-tenths, how much are they? |
| − | |style="text-align:right;"|ואם יאמר הוסף ג' שמיניות על ז' עשיריות כמה יהיו{{#annotend:tIym}} | + | :<math>\scriptstyle\frac{3}{8}+\frac{7}{10}</math> |
| + | |style="text-align:right;"|ואם יאמר הוסף ג' שמיניות על ז' עשיריות כמה יהיו{{#annotend:tIym}}‫<ref>ואם יאמר... כמה יהיו: MS Ithaca om.</ref> | ||
|- | |- | ||
| | | | ||
| − | :<span style=color: | + | :<span style=color:green>'''Common denominator:'''</span> Say: an eighth and a tenth are found in 8 times 10, which is 80. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{8\sdot10=80}}</math> |
| + | |style="text-align:right;"|אמור שמינית ועשירי' ימצאו בח' פעמים י' שהם פ‫' | ||
|- | |- | ||
| | | | ||
| + | :Add [its] 3-eighths, which is 30, to its 7-tenths, which is 56; the total is 86 parts of 80, which is 1 integer and 6 parts of 80. | ||
:<math>\scriptstyle{\color{blue}{\frac{3}{8}+\frac{7}{10}=\frac{\left(\frac{3}{8}\sdot80\right)+\left(\frac{7}{10}\sdot80\right)}{80}=\frac{30+56}{80}=\frac{86}{80}=1+\frac{6}{80}}}</math> | :<math>\scriptstyle{\color{blue}{\frac{3}{8}+\frac{7}{10}=\frac{\left(\frac{3}{8}\sdot80\right)+\left(\frac{7}{10}\sdot80\right)}{80}=\frac{30+56}{80}=\frac{86}{80}=1+\frac{6}{80}}}</math> | ||
| − | |style="text-align:right;"|ותוסיף ג' שמיניות והם ל' על ז' | + | |style="text-align:right;"|ותוסיף ג' שמיניות והם ל' על ז' עשרותיו והם נ"ו יהיו הכל פ"ו חלקים מפ' שהם א' שלם וו' חלקים מפ‫' |
|- | |- | ||
| | | | ||
| − | *{{#annot:⅔-³/₇|678|mJ1K}}<math>\scriptstyle\frac{2}{3}-\frac{3}{7}</math> | + | *{{#annot:⅔-³/₇|678|mJ1K}}If one says: subtract 3-sevenths from 2-thirds. |
| + | :<math>\scriptstyle\frac{2}{3}-\frac{3}{7}</math> | ||
|style="text-align:right;"|ואם יאמר השלך ג' שביעיות מב' שלישיות{{#annotend:mJ1K}} | |style="text-align:right;"|ואם יאמר השלך ג' שביעיות מב' שלישיות{{#annotend:mJ1K}} | ||
|- | |- | ||
| | | | ||
| − | :<span style=color: | + | :<span style=color:green>'''Common denominator:'''</span> Say: a third and a seventh are found in 3 times 7, which is 21. |
| + | :<math>\scriptstyle{\color{blue}{3\sdot7=21}}</math> | ||
|style="text-align:right;"|אמו' שלישית ושביעית ימצאו בג' פעמ' ז' שהם כ"א | |style="text-align:right;"|אמו' שלישית ושביעית ימצאו בג' פעמ' ז' שהם כ"א | ||
|- | |- | ||
| | | | ||
| + | :Take its 2-thirds, which is 14, and subtract from it its 3-sevenths, which is 9; 5 parts of 21 remain. | ||
:<math>\scriptstyle{\color{blue}{\frac{2}{3}-\frac{3}{7}=\frac{\left(\frac{2}{3}\sdot21\right)-\left(\frac{3}{7}\sdot21\right)}{21}=\frac{14-9}{21}=\frac{5}{21}}}</math> | :<math>\scriptstyle{\color{blue}{\frac{2}{3}-\frac{3}{7}=\frac{\left(\frac{2}{3}\sdot21\right)-\left(\frac{3}{7}\sdot21\right)}{21}=\frac{14-9}{21}=\frac{5}{21}}}</math> | ||
| − | |style="text-align:right;"|וקח ב' שלישיותיו והם י"ד ותשלך מהם ג' שביעיותיו והם ט' וישארו ה' חלקים מכ"א | + | |style="text-align:right;"|וקח ב' שלישיותיו והם י"ד ותשלך מהם ג' שביעיותיו והם ט' וישארו ה' חלקים מכ"א‫<ref>ואם יאמר השלך... חלקים מכ"א: MS Ithaca om.</ref> |
|- | |- | ||
| | | | ||
| − | *{{#annot:⅖-⅜|678|fRT6}}<math>\scriptstyle\frac{2}{5}-\frac{3}{8}</math> | + | *{{#annot:⅖-⅜|678|fRT6}}If one says: how much greater are 3-eighths than 2-fifths? |
| + | :<math>\scriptstyle\frac{2}{5}-\frac{3}{8}</math> | ||
|style="text-align:right;"|ואם יאמר כמה הם יותר ג' שמניות מב' חמשיות{{#annotend:fRT6}} | |style="text-align:right;"|ואם יאמר כמה הם יותר ג' שמניות מב' חמשיות{{#annotend:fRT6}} | ||
|- | |- | ||
| | | | ||
| − | :<span style=color: | + | :<span style=color:green>'''Common denominator:'''</span> Say: a fifth and an eighth are found in 5 times 8, which is 40. |
| + | :<math>\scriptstyle{\color{blue}{5\sdot8=40}}</math> | ||
|style="text-align:right;"|אמו' חמישיות ושמינית ימצא בה' פעמ' ח' שהם מ‫' | |style="text-align:right;"|אמו' חמישיות ושמינית ימצא בה' פעמ' ח' שהם מ‫' | ||
|- | |- | ||
| | | | ||
| + | :2-fifths are 16; 3-eighths are 15. We find that 2-fifths are greater that 3-eighths by one part of 40. | ||
:<math>\scriptstyle{\color{blue}{\frac{2}{5}-\frac{3}{8}=\frac{\left(\frac{2}{5}\sdot40\right)-\left(\frac{3}{8}\sdot40\right)}{40}=\frac{16-15}{40}=\frac{1}{40}}}</math> | :<math>\scriptstyle{\color{blue}{\frac{2}{5}-\frac{3}{8}=\frac{\left(\frac{2}{5}\sdot40\right)-\left(\frac{3}{8}\sdot40\right)}{40}=\frac{16-15}{40}=\frac{1}{40}}}</math> | ||
| − | |style="text-align:right;"|ב' חמישיות הם י"ו וג' שמיניות הם ט"ו נמצא שהב' חמישיות הם יותר מג' שמיניות חלק אחת ממ‫' | + | |style="text-align:right;"|ב' חמישיות הם י"ו וג' שמיניות הם ט"ו נמצא שהב' חמישיות הם יותר מג' שמיניות חלק אחת ממ'‫<ref>ואם יאמר כמה הם יותר...חלק אחת ממ': MS Ithaca om.</ref> |
|- | |- | ||
| | | | ||
| − | *{{#annot: | + | *{{#annot:⅜·⅖|668|8Loc}}If one says: how much are 3-eighths of 2-fifths? |
| + | :<math>\scriptstyle\frac{3}{8}\sdot\frac{2}{5}</math> | ||
|style="text-align:right;"|ואם יאמ' כמה הם ג' שמיניות מב' חמישיות{{#annotend:8Loc}} | |style="text-align:right;"|ואם יאמ' כמה הם ג' שמיניות מב' חמישיות{{#annotend:8Loc}} | ||
|- | |- | ||
| | | | ||
| − | :<span style=color: | + | :<span style=color:green>'''Common denominator:'''</span> Say: an eighth and a fifth are found in 5 times 8, which is 40. |
| + | :<math>\scriptstyle{\color{blue}{5\sdot8=40}}</math> | ||
|style="text-align:right;"|אמור שמינית וחמישית ימצאו בה' פע' ח' שהם מ‫' | |style="text-align:right;"|אמור שמינית וחמישית ימצאו בה' פע' ח' שהם מ‫' | ||
|- | |- | ||
| | | | ||
| + | :2-fifths of 40 are 16; 3-eighths of 16 are 6; so they are 6 parts of 40. | ||
:<math>\scriptstyle{\color{blue}{\frac{3}{8}\sdot\frac{2}{5}=\frac{\frac{3}{8}\sdot\left(\frac{2}{5}\sdot40\right)}{40}=\frac{\frac{3}{8}\sdot16}{40}=\frac{6}{40}}}</math> | :<math>\scriptstyle{\color{blue}{\frac{3}{8}\sdot\frac{2}{5}=\frac{\frac{3}{8}\sdot\left(\frac{2}{5}\sdot40\right)}{40}=\frac{\frac{3}{8}\sdot16}{40}=\frac{6}{40}}}</math> | ||
|style="text-align:right;"|וב' חמישיות מ' הם י"ו וג' שמיניות י"ו הם ו' א"כ הם ו' חלקי' ממ‫' | |style="text-align:right;"|וב' חמישיות מ' הם י"ו וג' שמיניות י"ו הם ו' א"כ הם ו' חלקי' ממ‫' | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:⅜÷⅖|552|gX0D}}<math>\scriptstyle\frac{3}{8}\div\frac{2}{5}</math> | + | *{{#annot:⅜÷⅖|552|gX0D}}If one says: divide 3-eighths by 2-fifths |
| + | :<math>\scriptstyle\frac{3}{8}\div\frac{2}{5}</math> | ||
|style="text-align:right;"|ואם יאמ' חלק ג' שמיניות על ב' חמשיות{{#annotend:gX0D}} | |style="text-align:right;"|ואם יאמ' חלק ג' שמיניות על ב' חמשיות{{#annotend:gX0D}} | ||
|- | |- | ||
| | | | ||
| − | :<span style=color: | + | :<span style=color:green>'''Common denominator:'''</span> Say: an eighth and a fifth are found in 5 times 8, which is 40. |
| + | :<math>\scriptstyle{\color{blue}{5\sdot8=40}}</math> | ||
|style="text-align:right;"|אמו' שמינית וחומש ימצאו בה' פעמ' ח' שהם מ‫' | |style="text-align:right;"|אמו' שמינית וחומש ימצאו בה' פעמ' ח' שהם מ‫' | ||
|- | |- | ||
| | | | ||
| + | :3-eighths of 40 are 15; its 2-fifths are 16; divide 15 by 16. | ||
:<math>\scriptstyle{\color{blue}{\frac{3}{8}\div\frac{2}{5}=\frac{\frac{3}{8}\sdot40}{\frac{2}{5}\sdot40}=\frac{15}{16}}}</math> | :<math>\scriptstyle{\color{blue}{\frac{3}{8}\div\frac{2}{5}=\frac{\frac{3}{8}\sdot40}{\frac{2}{5}\sdot40}=\frac{15}{16}}}</math> | ||
|style="text-align:right;"|וג' שמניות מ' הם ט"ו וב' חמשיותיו הם י"ו חלק ט"ו על י"ו | |style="text-align:right;"|וג' שמניות מ' הם ט"ו וב' חמשיותיו הם י"ו חלק ט"ו על י"ו | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:⅔×⅖|17|BRVP}}<math>\scriptstyle\frac{2}{3}\times\frac{2}{5}</math> | + | *{{#annot:⅔×⅖|17|BRVP}}If one says: multiply 2-thirds by 2-fifths. |
| + | :<math>\scriptstyle\frac{2}{3}\times\frac{2}{5}</math> | ||
|style="text-align:right;"|ואם יאמר חשוב ב' שלישים עם ב' חמישיות{{#annotend:BRVP}} | |style="text-align:right;"|ואם יאמר חשוב ב' שלישים עם ב' חמישיות{{#annotend:BRVP}} | ||
|- | |- | ||
| | | | ||
| + | :Multiply three by 5, which are the names of the parts; they are 15. | ||
| + | |style="text-align:right;"|תחשוב שלשה על ה' שהם שמות החלקים יהיו ט"ו | ||
| + | |- | ||
| + | | | ||
| + | :Say: 2 times 2, because they are the [numbers] of the parts; they are 4. | ||
| + | |style="text-align:right;"|ואמור ב' פעמ' ב' כי ב' הם שמות החלקים ויהיו ד‫' | ||
| + | |- | ||
| + | | | ||
| + | :So, 2-thirds by 2-fifths are 4 parts of 15. | ||
:<math>\scriptstyle{\color{blue}{\frac{2}{3}\times\frac{2}{5}=\frac{2\sdot2}{3\sdot5}=\frac{4}{15}}}</math> | :<math>\scriptstyle{\color{blue}{\frac{2}{3}\times\frac{2}{5}=\frac{2\sdot2}{3\sdot5}=\frac{4}{15}}}</math> | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|הרי שב' שלישיות על ב' חמישיות הם ד' חלקים מט"ו מן האחד |
|- | |- | ||
| | | | ||
| − | *{{#annot:²/₁₁ײ/₁₃|17|md7k}}<math>\scriptstyle\frac{2}{11}\times\frac{2}{13}</math> | + | *{{#annot:²/₁₁ײ/₁₃|17|md7k}}If one says: multiply 2 parts of 11 by 2 parts of 13. |
| + | :<math>\scriptstyle\frac{2}{11}\times\frac{2}{13}</math> | ||
|style="text-align:right;"|ואם יאמר חשוב ב' חלקים מי"א באחד על ב' חלקים מי"ג מן האחד{{#annotend:md7k}} | |style="text-align:right;"|ואם יאמר חשוב ב' חלקים מי"א באחד על ב' חלקים מי"ג מן האחד{{#annotend:md7k}} | ||
|- | |- | ||
| | | | ||
| + | :Multiply the names of the parts, which are 11 by 13; the result is 143. | ||
| + | |style="text-align:right;"|תחשוב שמות החלקים והם י"א על י"ג שעולים קמ"ג | ||
| + | |- | ||
| + | | | ||
| + | :Multiply the numbers of the parts, which are 2; say: 2 times 2 is 4. | ||
| + | |style="text-align:right;"|ותרבה מספר החלקים שהם ב' ואמור ב' פעמים ב' ד‫' | ||
| + | |- | ||
| + | | | ||
| + | :So, the result is 4 parts of 143. | ||
:<math>\scriptstyle{\color{blue}{\frac{2}{11}\times\frac{2}{13}=\frac{2\sdot2}{11\sdot13}=\frac{4}{143}}}</math> | :<math>\scriptstyle{\color{blue}{\frac{2}{11}\times\frac{2}{13}=\frac{2\sdot2}{11\sdot13}=\frac{4}{143}}}</math> | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|הרי שעולים ד' חלקים מקמ"ג בא‫' |
|- | |- | ||
| | | | ||
| − | *{{#annot:⅖×⅒×⅔|17|q3mD}}<math>\scriptstyle\frac{2}{5}\times\frac{1}{10}\times\frac{2}{3}</math> | + | *{{#annot:⅖×⅒×⅔|17|q3mD}}If one says: how much are 2 parts of 5 by one-tenth by 2-thirds? |
| + | :<math>\scriptstyle\frac{2}{5}\times\frac{1}{10}\times\frac{2}{3}</math> | ||
|style="text-align:right;"|ואם יאמ' ב' חלקים מה' בעשירית האחד בב' שלישי האחר כמה הם{{#annotend:q3mD}} | |style="text-align:right;"|ואם יאמ' ב' חלקים מה' בעשירית האחד בב' שלישי האחר כמה הם{{#annotend:q3mD}} | ||
|- | |- | ||
| | | | ||
| + | :Know that the name of one [fraction] is fifty, which is five by ten. Multiply this 50 by 3, which is the name of the other fraction; the total is 150. | ||
| + | |style="text-align:right;"|דע כי שם האחד הם חמשים בעניין חמשה בעשרה ותמנה אלו הנ' בג' שהוא שם החלק השני ויהיה הכל ק"נ | ||
| + | |- | ||
| + | | | ||
| + | :Say: 2 times 2 is 4, because 2 is the number of the fractions. | ||
| + | |style="text-align:right;"|ואמור ב' פעמ' ב' ד' כי ב' הוא מספר החלקי‫' | ||
| + | |- | ||
| + | | | ||
| + | :So, this product is 4 parts of 150. | ||
:<math>\scriptstyle{\color{blue}{\frac{2}{5}\times\frac{1}{10}\times\frac{2}{3}=\frac{2\sdot2}{\left(5\sdot10\right)\sdot3}=\frac{4}{50\sdot3}=\frac{4}{150}}}</math> | :<math>\scriptstyle{\color{blue}{\frac{2}{5}\times\frac{1}{10}\times\frac{2}{3}=\frac{2\sdot2}{\left(5\sdot10\right)\sdot3}=\frac{4}{50\sdot3}=\frac{4}{150}}}</math> | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|הרי שהחשבו' הזה הוא ד' חלקי' מק"נ מהאחר |
|- | |- | ||
| | | | ||
| − | *{{#annot:⅖×⅒ײ/₈×⅓|17|aUsg}}<math>\scriptstyle\frac{2}{5}\times\frac{1}{10}\times\frac{2}{8}\times\frac{1}{3}</math> | + | *{{#annot:⅖×⅒ײ/₈×⅓|17|aUsg}}If one says: 2 parts of five by one-tenth by two parts of eight by one-third. |
| + | :<math>\scriptstyle\frac{2}{5}\times\frac{1}{10}\times\frac{2}{8}\times\frac{1}{3}</math> | ||
|style="text-align:right;"|ואם יאמ' ב' חלקים מחמשה בעשירית האחד בשני חלקים משמנה בשלישית האחד{{#annotend:aUsg}} | |style="text-align:right;"|ואם יאמ' ב' חלקים מחמשה בעשירית האחד בשני חלקים משמנה בשלישית האחד{{#annotend:aUsg}} | ||
|- | |- | ||
| | | | ||
| + | :You need to know the names of the fractions: | ||
| + | |style="text-align:right;"|אתה צריך לדעת את שמות החלקים | ||
| + | |- | ||
| + | | | ||
| + | :Multiply 5 by 10; it is 50 and this is the name of one fraction. | ||
| + | |style="text-align:right;"|ותחשוב ה' בי' והם נ' והוא שם החלק האחד | ||
| + | |- | ||
| + | | | ||
| + | :Multiply 8 by 3; it is 24 and this is the name of the other fraction. | ||
| + | |style="text-align:right;"|ותחשוב עוד ח' בג' ויהיו כ"ד והוא שם החלק השני | ||
| + | |- | ||
| + | | | ||
| + | :Then, multiply 50 by 24; it is one thousand and two hundred. | ||
| + | |style="text-align:right;"|וחשוב אחר כן נ' על כ"ד ויהיה אלף ומאתים | ||
| + | |- | ||
| + | | | ||
| + | :Say: 2 times 2; i.e. 4. | ||
| + | |style="text-align:right;"|ואמו' אחר כן ב' פעמ' ב' ר"ל ד‫' | ||
| + | |- | ||
| + | | | ||
| + | :So, they are 4 parts of one thousand and two hundred. | ||
:<math>\scriptstyle{\color{blue}{\frac{2}{5}\times\frac{1}{10}\times\frac{2}{8}\times\frac{1}{3}=\frac{2\sdot2}{\left(5\sdot10\right)\sdot\left(8\sdot3\right)}=\frac{4}{50\sdot24}=\frac{4}{1200}}}</math> | :<math>\scriptstyle{\color{blue}{\frac{2}{5}\times\frac{1}{10}\times\frac{2}{8}\times\frac{1}{3}=\frac{2\sdot2}{\left(5\sdot10\right)\sdot\left(8\sdot3\right)}=\frac{4}{50\sdot24}=\frac{4}{1200}}}</math> | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|הרי שהם ד' חלקים מאלף ומאתים מן האחר‫<ref>ואם יאמר חלק ג'... ומאתים מן האחר: MS Ithaca om.</ref> |
|- | |- | ||
| | | | ||
| − | === Multiplication of fractions === | + | === <span style=color:green>Multiplication of fractions</span> === |
| | | | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:⅓×1|17|dp1H}}<math>\scriptstyle\frac{1}{3}\times1=\frac{1}{3}</math> | + | *{{#annot:⅓×1|17|dp1H}}When we multiply the third by one, it is a third. |
| − | |style="text-align:right;"|כשנרבה השליש באחד הוא שליש{{#annotend:dp1H}} | + | :<math>\scriptstyle\frac{1}{3}\times1=\frac{1}{3}</math> |
| + | |style="text-align:right;"|<big>כשנרבה השליש באחד</big> הוא שליש{{#annotend:dp1H}} | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:⅓×2|17|CmHn}}<math>\scriptstyle\frac{1}{3}\times2=\frac{2}{3}</math> | + | *{{#annot:⅓×2|17|CmHn}}When we multiply the third by two it is 2-thirds. |
| + | :<math>\scriptstyle\frac{1}{3}\times2=\frac{2}{3}</math> | ||
|style="text-align:right;"|וכשנרבה השליש בשנים הוא ב' שלישי האחד{{#annotend:CmHn}} | |style="text-align:right;"|וכשנרבה השליש בשנים הוא ב' שלישי האחד{{#annotend:CmHn}} | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:⅓×⅓|17|p54W}}<math>\scriptstyle\frac{1}{3}\times\frac{1}{3}=\frac{1}{3}\sdot\frac{1}{3}</math> | + | *{{#annot:⅓×⅓|17|p54W}}When we multiply a third by a third, it is a third of a third. |
| + | :<math>\scriptstyle\frac{1}{3}\times\frac{1}{3}=\frac{1}{3}\sdot\frac{1}{3}</math> | ||
|style="text-align:right;"|וכשנרבה שליש בשליש הוא שליש בשליש{{#annotend:p54W}} | |style="text-align:right;"|וכשנרבה שליש בשליש הוא שליש בשליש{{#annotend:p54W}} | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:¼×¼|17|q5Pm}}<math>\scriptstyle\frac{1}{4}\times\frac{1}{4}=\frac{1}{4}\sdot\frac{1}{4}</math> | + | *{{#annot:¼×¼|17|q5Pm}}The quarter by a quarter is a quarter of a quarter. |
| + | :<math>\scriptstyle\frac{1}{4}\times\frac{1}{4}=\frac{1}{4}\sdot\frac{1}{4}</math> | ||
|style="text-align:right;"|והרביע ברביע הוא רביע הרביע{{#annotend:q5Pm}} | |style="text-align:right;"|והרביע ברביע הוא רביע הרביע{{#annotend:q5Pm}} | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:⅕×⅕|17|GtWm}}<math>\scriptstyle\frac{1}{5}\times\frac{1}{5}=\frac{1}{5}\sdot\frac{1}{5}</math> | + | *{{#annot:⅕×⅕|17|GtWm}}The fifth by a fifth is a fifth of a fifth. |
| − | |style="text-align:right;"|וחומש בחומש הוא חומ' החומש | + | :<math>\scriptstyle\frac{1}{5}\times\frac{1}{5}=\frac{1}{5}\sdot\frac{1}{5}</math> |
| + | |style="text-align:right;"|וחומש בחומש הוא חומ' החומש{{#annotend:GtWm}} | ||
| + | |- | ||
| + | | | ||
| + | :And so on. | ||
| + | |style="text-align:right;"|וכן כלם | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:⅔×⅔|17|Nlzy}}<math>\scriptstyle\frac{2}{3}\times\frac{2}{3}</math> | + | *{{#annot:⅔×⅔|17|Nlzy}}If we multiply 2-thirds by 2-thirds. |
| + | :<math>\scriptstyle\frac{2}{3}\times\frac{2}{3}</math> | ||
|style="text-align:right;"|ואם נרבה ב' שלישי בב' שלישי{{#annotend:Nlzy}} | |style="text-align:right;"|ואם נרבה ב' שלישי בב' שלישי{{#annotend:Nlzy}} | ||
|- | |- | ||
| | | | ||
| + | :Say: 3 times 3 is 9. | ||
| + | |style="text-align:right;"|אמור ג' פעמים [.] ג' ט‫' | ||
| + | |- | ||
| + | | | ||
| + | :Then, say: 2 times 2 is 4. | ||
| + | |style="text-align:right;"|ואחר כך אמור ב' פעמים ב' פעמים הרי ד‫' | ||
| + | |- | ||
| + | | | ||
| + | :So, if we multiply 2-thirds by 2-thirds, they are 4 parts of 9. | ||
:<math>\scriptstyle{\color{blue}{\frac{2}{3}\times\frac{2}{3}=\frac{2\sdot2}{3\sdot3}=\frac{4}{9}}}</math> | :<math>\scriptstyle{\color{blue}{\frac{2}{3}\times\frac{2}{3}=\frac{2\sdot2}{3\sdot3}=\frac{4}{9}}}</math> | ||
| − | |style="text-align:right;"| | + | |style="text-align:right;"|הרי שאם נרבה ב' שלישי על ב' שלישי הם ד' חלקי' מט' באחד |
|- | |- | ||
| | | | ||
| − | === Proportions with fractions === | + | === <span style=color:green>Proportions with fractions</span> === |
| | | | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:⅓÷¼=⅕÷X|567|D0xp}}<math>\scriptstyle\frac{1}{3}:\frac{1}{4}=\frac{1}{5}:X</math> | + | *{{#annot:⅓÷¼=⅕÷X|567|D0xp}}When a man says: if a third is worth a quarter, how much is a fifth worth according to this calculation? |
| + | :<math>\scriptstyle\frac{1}{3}:\frac{1}{4}=\frac{1}{5}:X</math> | ||
|style="text-align:right;"|אם יאמר אדם אם שליש שוה רביע החומש כפי זה החשבון כמה שוה{{#annotend:D0xp}} | |style="text-align:right;"|אם יאמר אדם אם שליש שוה רביע החומש כפי זה החשבון כמה שוה{{#annotend:D0xp}} | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Common denominator:'''</span> Say: a third, a quarter and a fifth are found in 60. | |
|style="text-align:right;"|אמור שליש ורביע וחומש ימצאו בס‫' | |style="text-align:right;"|אמור שליש ורביע וחומש ימצאו בס‫' | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\frac{1}{3}\sdot60=20}}</math> | + | :The third of 60 is 20. |
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{3}\sdot60=20}}</math> | ||
|style="text-align:right;"|השליש מס' הוא כ‫' | |style="text-align:right;"|השליש מס' הוא כ‫' | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\frac{1}{4}\sdot60=15}}</math> | + | :The quarter is 15. |
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{4}\sdot60=15}}</math> | ||
|style="text-align:right;"|א"כ הרביע הוא ט"ו | |style="text-align:right;"|א"כ הרביע הוא ט"ו | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\frac{1}{5}\sdot60=12}}</math> | + | :The fifth is 12. |
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{5}\sdot60=12}}</math> | ||
|style="text-align:right;"|והחומש הוא י"ב | |style="text-align:right;"|והחומש הוא י"ב | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> Say: if 20, which is one-third of 60, is worth 15, which is the quarter, how much is the fifth, which is 12, worth? | |
| + | :<math>\scriptstyle{\color{blue}{20:15=12:\left(X\sdot60\right)}}</math> | ||
|style="text-align:right;"|ואמור אם כ' שהוא שליש מס' שוה ט"ו שהוא הרביע החומש שהוא י"ב כמה שוה | |style="text-align:right;"|ואמור אם כ' שהוא שליש מס' שוה ט"ו שהוא הרביע החומש שהוא י"ב כמה שוה | ||
|- | |- | ||
| | | | ||
| − | :: | + | :Say: 12 times 15 is 180 parts. |
| − | |style="text-align:right;"|אמור י"ב פעמי' ט"ו הם ק"פ חלקים | + | |style="text-align:right;"|אמור י"ב פעמי' ט"ו הם ק"פ חלקים |
|- | |- | ||
| | | | ||
| − | + | :Divide it by 20; the result is 9 and they are 9 parts of 60. | |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{X=\frac{\frac{12\sdot15}{20}}{60}=\frac{\frac{180}{20}}{60}=\frac{9}{60}}}</math> |
| + | |style="text-align:right;"|חלקם בכ' יבאו ט' והם ט' חלקים מס' באחד | ||
|- | |- | ||
| | | | ||
| + | *{{#annot:3⅓÷4¼=5⅕÷X|567|JsBk}}If one says: if 3 and a third equals 4 and a quarter, how much is 5 and a fifth equal to? | ||
| + | :<math>\scriptstyle\left(3+\frac{1}{3}\right):\left(4+\frac{1}{4}\right)=\left(5+\frac{1}{5}\right):X</math> | ||
| + | |style="text-align:right;"|<big>אם יאמר אדם</big> אם ג' ושליש שוים ד' ורביע ה' [ו]חומש כמה שוים{{#annotend:JsBk}} | ||
| + | |- | ||
| + | | | ||
| + | :Say: 5 and a fifth times 4 and a quarter, then divide it by 3 and a third. This is the procedure: | ||
:<math>\scriptstyle{\color{blue}{X=\frac{\left(5+\frac{1}{5}\right)\sdot\left(4+\frac{1}{4}\right)}{3+\frac{1}{3}}}}</math> | :<math>\scriptstyle{\color{blue}{X=\frac{\left(5+\frac{1}{5}\right)\sdot\left(4+\frac{1}{4}\right)}{3+\frac{1}{3}}}}</math> | ||
|style="text-align:right;"|אמור ה' וחומש פעמים ד' ורביע וחלקם בג' ושליש וזה סדר עשייתו | |style="text-align:right;"|אמור ה' וחומש פעמים ד' ורביע וחלקם בג' ושליש וזה סדר עשייתו | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{5+\frac{1}{5}=\frac{\left(5\sdot5\right)+1}{5}=\frac{25+1}{5}=\frac{26}{5}}}</math> | + | :Take 5 and a fifth and say: 5 times 5 is 25; plus one fifth, it is 26. |
| − | |style="text-align:right;"|תפוש ה' וחומש ואמור ה' פעמים ה' הם כ"ה וא' חומש הרי כ"ו | + | :<math>\scriptstyle{\color{blue}{5+\frac{1}{5}=\frac{\left(5\sdot5\right)+1}{5}=\frac{25+1}{5}=\frac{26}{5}}}</math> |
| + | |style="text-align:right;"|תפוש ה' וחומש ואמור ה' פעמים ה' הם‫<ref>ה' הם: MS Ithaca om.</ref> כ"ה וא' חומש הרי כ"ו | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{4+\frac{1}{4}=\frac{\left(4\sdot4\right)+1}{4}=\frac{16+1}{4}=\frac{17}{4}}}</math> | + | :Then, take 4 and a quarter and say: 4 times 4 is 16; plus one quarter, it is 17. |
| + | :<math>\scriptstyle{\color{blue}{4+\frac{1}{4}=\frac{\left(4\sdot4\right)+1}{4}=\frac{16+1}{4}=\frac{17}{4}}}</math> | ||
|style="text-align:right;"|אחר זה תפוש ד' ורביע ואמור ד' פעמים ד' י"ו וא' רביע הרי י"ז | |style="text-align:right;"|אחר זה תפוש ד' ורביע ואמור ד' פעמים ד' י"ו וא' רביע הרי י"ז | ||
|- | |- | ||
| | | | ||
| − | : | + | :Multiply 17 by 26; the result is 442. |
| − | |style="text-align:right;"|ותרבה י"ז עם כ"ו ויעלו תמ"ב | + | |style="text-align:right;"|ותרבה י"ז עם כ"ו ויעלו תמ"ב |
|- | |- | ||
| | | | ||
| − | : | + | :We divide it by 4 times 5, which is 20; the result is 22 and a tenth. |
| − | + | :<math>\scriptstyle{\color{blue}{\left(5+\frac{1}{5}\right)\sdot\left(4+\frac{1}{4}\right)=\frac{26}{5}\sdot\frac{17}{4}=\frac{26\sdot17}{5\sdot4}=\frac{442}{20}=22+\frac{1}{10}}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ונחלק אותם בד' פעמים ה' שהם כ' יבואו כ"ב וא' עשירי‫' |
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{3+\frac{1}{3}=\frac{\left(3\sdot3\right)+1}{3}=\frac{9+1}{3}=\frac{10}{3}}}</math> | + | :Since we need to divide it by 3 and a third, convert 22 and a tenth into thirds. Say: 3 times 22 is 66; 3 times a tenth is 3-tenths. So, it is 66 and 3-tenths. |
| + | :<math>\scriptstyle{\color{blue}{\left(5+\frac{1}{5}\right)\sdot\left(4+\frac{1}{4}\right)=22+\frac{1}{10}=\frac{3\sdot\left(22+\frac{1}{10}\right)}{3}=\frac{66+\frac{3}{10}}{3}}}</math> | ||
| + | |style="text-align:right;"|ובעבור שנצטרך לחלקם לג' ושליש עשה מכ"ב וא' עשירית כולם שלישיות ואמור ג"פ כ"ב ס"ו וג' פעמי' א' עשירית הרי ג' עשיריות הרי ס"ו וג' עשיריות | ||
| + | |- | ||
| + | | | ||
| + | :We need to divide this by 3 and a third. The procedure is that you convert 3 and a third into thirds and say: 3 times 3 is 9; plus 1, it is 10. | ||
| + | :<math>\scriptstyle{\color{blue}{3+\frac{1}{3}=\frac{\left(3\sdot3\right)+1}{3}=\frac{9+1}{3}=\frac{10}{3}}}</math> | ||
|style="text-align:right;"|וצריך שנחלק אותם בג' ושליש וסדר חילופו שתעשה מג' ושליש כלם שלישיות ואמור ג'פ'ג' ט' וא' הרי י‫' | |style="text-align:right;"|וצריך שנחלק אותם בג' ושליש וסדר חילופו שתעשה מג' ושליש כלם שלישיות ואמור ג'פ'ג' ט' וא' הרי י‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | :If we divide 66 into 10 parts, the result is 6 and 6-tenths. |
| − | |style="text-align:right;"|ואם נחלק ס"ו בי' חלקים יבואו ו' וו' עשיריות | + | |style="text-align:right;"|ואם נחלק ס"ו בי' חלקים יבואו ו' וו' עשיריות |
| − | נחלק אח"כ ג' עשיריות בי' חלקי' ישאר ג' חלקי' ממאה< | + | |- |
| − | הרי שעולה החשבון ו' וו' עשיריות וג' חלקי' ממאה< | + | | |
| − | וכדי שנוכל לקבץ הכל יחד ו' עשיריות חלקים ממאה ויהיו ס' חלקים מק' וג' חלקים מק' שהיו בידינו הרי ס"ג | + | :Next, we divide 3-tenths into 10 parts; 3 parts of a hundred remain. |
| − | תמצא מדברינו שאם ג' ושליש שוי' ד' ורביע ה' וחומש שוי' ו' וס"ג חלקים ממאה | + | |style="text-align:right;"|נחלק אח"כ ג' עשיריות בי' חלקי' ישאר ג' חלקי' ממאה‫<ref>ממאה: MS Ithaca ומאה</ref> |
| + | |- | ||
| + | | | ||
| + | :So, the result is 6, 6-tenths and 3 parts of a hundred. | ||
| + | |style="text-align:right;"|הרי שעולה החשבון ו' וו' עשיריות וג' חלקי' ממאה‫<ref>ממאה: MS Ithaca ומאה</ref> | ||
| + | |- | ||
| + | | | ||
| + | :In order that we can sum it all up, [we convert] 6-tenths [into] parts of a hundred; this is 60 parts of 100; plus the 3 parts of 100 that we had, this is 63. | ||
| + | |style="text-align:right;"|וכדי שנוכל לקבץ הכל יחד ו' עשיריות חלקים ממאה ויהיו ס' חלקים מק' וג' חלקים מק' שהיו בידינו הרי ס"ג | ||
| + | |- | ||
| + | | | ||
| + | :You find from our words that if 3 and a third equals 4 and a quarter, then 5 and a fifth equals 6 and 63 parts of a hundred. | ||
| + | |style="text-align:right;"|תמצא מדברינו שאם ג' ושליש שוי' ד' ורביע ה' וחומש שוי' ו' וס"ג חלקים ממאה | ||
|- | |- | ||
| − | |} | + | | colspan="2"| |
| − | { | + | :<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle X&\scriptstyle=\frac{\left(5+\frac{1}{5}\right)\sdot\left(4+\frac{1}{4}\right)}{3+\frac{1}{3}}=\frac{\frac{66+\frac{3}{10}}{3}}{\frac{10}{3}}=\frac{66+\frac{3}{10}}{10}=\frac{66}{10}+\frac{\frac{3}{10}}{10}=6+\frac{6}{10}+\frac{3}{100}\\&\scriptstyle=6+\frac{60}{100}+\frac{3}{100}=6+\frac{63}{100}\\\end{align}}}</math> |
|- | |- | ||
| | | | ||
| − | == Word Problems == | + | == <span style=color:green>Word Problems</span> == |
| | | | ||
|- | |- | ||
| | | | ||
| − | === Exchange Problems === | + | === <span style=color:green>Exchange Problems</span> === |
| | | | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:two currencies|632|YdMT}}If 7 of Pisa are worth 9 of Cortona, how many of Cortona are 100 of Pisa worth? | + | *{{#annot:two currencies|632|YdMT}}If a man says: 7 of Pisa are worth 9 of Cortona, how many liṭra of Cortona are 100 liṭra of Pisa worth? |
:<math>\scriptstyle\frac{7}{9}=\frac{100}{X}</math> | :<math>\scriptstyle\frac{7}{9}=\frac{100}{X}</math> | ||
|style="text-align:right;"|אם יאמר אדם ז' פיסאני שוים ט' קונטוניסי<br> | |style="text-align:right;"|אם יאמר אדם ז' פיסאני שוים ט' קונטוניסי<br> | ||
| Line 2,732: | Line 3,671: | ||
|- | |- | ||
| | | | ||
| − | :: | + | :Do it this way: |
| − | |style="text-align:right;"|עשה על זה הדרך | + | |style="text-align:right;"|עשה על זה הדרך |
|- | |- | ||
| | | | ||
| − | : | + | :It is known that if 7 pisani are worth 9 cortonisi, then 7 dinar of Pisa are worth 9 dinar of Cortona; 7 liṭra of Pisa are worth 9 liṭra of Cortona; and 700 liṭra of Pisa are worth 900 liṭra of Cortona; and the same for any currency you want. |
| − | |style="text-align:right;"|ז' דינ' פיסאני שוים ט' דנרי' קוטורניסי | + | |style="text-align:right;"|ידוע כי אם ז' פיסאני שוים ט' קוטורניסי ז' דינ' פיסאני שוים ט' דנרי' קוטורניסי וז' ליט' פיסאני שוים ט' ליט' קוטורניסי ות"ש ליט' פיסאני שוים תת"ק ליט' קוטורניסי וכן לכל מטבע שתרצה |
|- | |- | ||
| | | | ||
| − | ::: | + | :<span style=color:green>'''Rule of Three:'''</span> So, this question is as if it is asked: If 700 liṭra of Pisa are worth 900 liṭra of Cortona, how much are 100 liṭra of Pisa worth? |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{700:900=100:X}}</math> |
| + | |style="text-align:right;"|א"כ השאלה הזאת היא כאלו שאל אם ת"ש ליט' פיסאני שוים תת"ק ליט' קוטורניסי ק' ליט' פיסאני כמה שוים | ||
|- | |- | ||
| | | | ||
| − | ::: | + | :<span style=color:green>'''Rule of Three:'''</span> To make the calculation easier we say: if 7 is equal to 9, how much is 100 equal to? |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{7:9=100:X}}</math> |
| − | + | |style="text-align:right;"|ולהקל החשבון נאמר אם ז' שוים ט' ק' כמה שוים | |
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | :: | + | :Say: 100 times 9 is 900. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אמור ק' פעמי' ט' תת"ק |
|- | |- | ||
| | | | ||
| − | : | + | :Divide it by 7; it is 128 liṭra, 11 dinar and [3-sevenths]; and this number of Cortona is equal to 100 of Pisa. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{X=\frac{100\sdot9}{7}=\frac{900}{7}=128+\frac{11}{20}+\frac{\frac{3}{7}}{20}}}</math> |
| + | |style="text-align:right;"|חלקם בז' יהיו קכ"ח ליט' וי"א דינ' וא' שביע וכן ישוו הק' ליט' פיסא' לקוטורניסי | ||
|- | |- | ||
| | | | ||
| − | + | *{{#annot:two currencies|632|ANcb}}If a man says: if 5 and a quarter of Pisa are worth 7 of Cortona, how many liṭra of Cortona are a thousand liṭra of Pisa worth? | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | *{{#annot:two currencies|632|ANcb}}If | ||
:<math>\scriptstyle\frac{5+\frac{1}{4}}{7}=\frac{1000}{X}</math> | :<math>\scriptstyle\frac{5+\frac{1}{4}}{7}=\frac{1000}{X}</math> | ||
|style="text-align:right;"|אם יאמר אדם אם ה' פיסאני ורביע שוים ז' קוטורניסי<br> | |style="text-align:right;"|אם יאמר אדם אם ה' פיסאני ורביע שוים ז' קוטורניסי<br> | ||
| Line 2,770: | Line 3,704: | ||
|- | |- | ||
| | | | ||
| − | : | + | :You see that this calculation has a fraction in one part and this fraction is a quarter. |
| − | |style="text-align:right;"|הנך רואה כי זה החשבון הוא שבור מחלק אחד וזה השבור הוא רביע | + | |style="text-align:right;"|הנך רואה כי זה החשבון הוא שבור מחלק אחד וזה השבור הוא רביע |
| − | |||
|- | |- | ||
| | | | ||
| − | :::<math>\scriptstyle{\color{blue}{4\sdot\left(5+\frac{1}{4}\right)=21}}</math> | + | :Therefore, we multiply both parts, which are 5 and a quarter and 7, by 4, i.e. we convert all into quarters. |
| + | |style="text-align:right;"|לכן נרבה הב' חלקים שהם ה' ורביע וז' בד' דהיינו שנעשה כלם רביעיים | ||
| + | |- | ||
| + | | | ||
| + | :Say: 4 times 5 pisani and a quarter; the result is 21 pisani. | ||
| + | :<math>\scriptstyle{\color{blue}{4\sdot\left(5+\frac{1}{4}\right)=21}}</math> | ||
|style="text-align:right;"|ואמ' ד' פעמי' ה' פיסאני ורביע יבואו כ"א פיסאני | |style="text-align:right;"|ואמ' ד' פעמי' ה' פיסאני ורביע יבואו כ"א פיסאני | ||
|- | |- | ||
| | | | ||
| − | : | + | :4 times 7 cortonisi; the result is 28 cortonisi. |
| + | :<math>\scriptstyle{\color{blue}{4\sdot7=28}}</math> | ||
|style="text-align:right;"|וד' פעמי' ז' קוטורניסי יבואו כ"ח קוטורניסי | |style="text-align:right;"|וד' פעמי' ז' קוטורניסי יבואו כ"ח קוטורניסי | ||
|- | |- | ||
| | | | ||
| − | : | + | :So, 21 pisani are worth 28 cortonosi. |
|style="text-align:right;"|הרי שכ"א פיסאני שוים כ"ח קוטורניסי | |style="text-align:right;"|הרי שכ"א פיסאני שוים כ"ח קוטורניסי | ||
|- | |- | ||
| | | | ||
| − | : | + | :To make the case easier, we reduce them by seven, because both parts have a seventh. |
|style="text-align:right;"|ולהקל הענין נחלקם בשביע בעבור שבשני החלקים ימצא שביע | |style="text-align:right;"|ולהקל הענין נחלקם בשביע בעבור שבשני החלקים ימצא שביע | ||
|- | |- | ||
| | | | ||
| − | : | + | :The seventh of 21 is 3. |
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{7}\sdot21=3}}</math> | ||
|style="text-align:right;"|השביע מכ"א הוא ג‫' | |style="text-align:right;"|השביע מכ"א הוא ג‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | :The seventh of 28 is 4. |
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{7}\sdot28=4}}</math> | ||
|style="text-align:right;"|והשביע מכ"ח הוא ד‫' | |style="text-align:right;"|והשביע מכ"ח הוא ד‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | :We find that 3 pisani are worth 4 cortonisi. |
|style="text-align:right;"|נמצא שג' פיסאני שוים ד' קוטורניסי | |style="text-align:right;"|נמצא שג' פיסאני שוים ד' קוטורניסי | ||
|- | |- | ||
| | | | ||
| − | ::<span style=color: | + | :We want to know how much are a thousand liṭra of Pisa worth? |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואנו רוצים לדעת כמה שוים אלף ליט' פיסאני |
| − | אמור א"כ אם ג' פיסאני שוים ד' קוטורניסי אלף ליט' פיסאני כמה שוים | + | |- |
| + | | | ||
| + | :<span style=color:green>'''Rule of Three:'''</span> Say: if 3 pisani are worth 4 cortonisi, how much are a thousand liṭra worth? | ||
| + | :<math>\scriptstyle{\color{blue}{3:4=1000:X}}</math> | ||
| + | |style="text-align:right;"|אמור א"כ אם ג' פיסאני שוים ד' קוטורניסי אלף ליט' פיסאני כמה שוים | ||
| + | |- | ||
| + | | | ||
| + | :Say: a thousand times 4 are 4 thousand. | ||
| + | |style="text-align:right;"|אמור אלף פעמי' ד' יבואו ד' אלפים | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{X=\frac{1000\sdot4}{3}=\frac{4000}{3}=1333+\frac{6}{20}+\frac{\frac{8}{12}}{20}}}</math> | + | :Divide them by 3; the result is 1333 [liṭra], 6 dinar and 8 pešuṭim and so are a thousand liṭra of Pisa worth in Cortona. |
| − | + | :<math>\scriptstyle{\color{blue}{X=\frac{1000\sdot4}{3}=\frac{4000}{3}=1333+\frac{6}{20}+\frac{\frac{8}{12}}{20}}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|חלקם בג' יבואו אלף ושל"ג וו' די' וח' פשוטי' וכן שוים אלף ליט' פיסאני לקוטרניסי‫<ref>אם יאמר אדם ז' פיסאני... אלף ליט' פיסאני לקוטרניסי: MS Ithaca om.</ref> |
| − | חלקם בג' יבואו אלף ושל"ג וו' די' וח' פשוטי' וכן שוים אלף ליט' פיסאני לקוטרניסי | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:two currencies|632|QWOi}}If | + | *{{#annot:two currencies|632|QWOi}}If a man says: if 7 silver ounces and a third are worth 73 liṭra and a quarter, how much are 19 silver ounces worth? |
:<math>\scriptstyle\frac{7+\frac{1}{3}}{73+\frac{1}{4}}=\frac{19}{X}</math> | :<math>\scriptstyle\frac{7+\frac{1}{3}}{73+\frac{1}{4}}=\frac{19}{X}</math> | ||
| − | |style="text-align:right;"|ואם יאמר אדם אם ז' אונקיו' ושליש מכסף שוי' ע"ג ליטרי' ורביע<br> | + | |style="text-align:right;"|‫<ref>37v</ref><big>ואם יאמר</big> אדם אם ז' אונקיו' ושליש מכסף שוי' ע"ג ליטרי' ורביע<br> |
י"ט אונקיו' מכסף כמה ישוו לפי חשבון זה{{#annotend:QWOi}} | י"ט אונקיו' מכסף כמה ישוו לפי חשבון זה{{#annotend:QWOi}} | ||
|- | |- | ||
| | | | ||
| − | :: | + | :Do this way: the fractions in this calculation are a third and a quarter; a third and a quarter are found in 12. |
| − | |style="text-align:right;"|עשה על זה הדרך השברי' שהם בזה החשבון הם שליש ורביע ושליש ורביע ימצאו בי"ב | + | |style="text-align:right;"|עשה על זה הדרך השברי' שהם בזה החשבון הם שליש ורביע ושליש ורביע ימצאו בי"ב |
| − | לכן יש לנו לרבות הב' חלקי' שהם ז' ושליש וע"ג ורביע בי"ב דהיינו לעשות מכלם חלקים מי"ב | + | |- |
| + | | | ||
| + | :Therefore, we have to multiply both parts, which are 7 and a third and 73 and a quarter, by 12, i.e. to convert all of them into parts of 12. | ||
| + | |style="text-align:right;"|לכן יש לנו לרבות הב' חלקי' שהם ז' ושליש וע"ג ורביע בי"ב דהיינו לעשות מכלם חלקים מי"ב | ||
|- | |- | ||
| | | | ||
| − | :::<math>\scriptstyle{\color{blue}{12\sdot\left(7+\frac{1}{3}\right)=88}}</math> | + | :Say: 12 times 7 and a third; the result is 88 silver ounces. |
| + | :<math>\scriptstyle{\color{blue}{12\sdot\left(7+\frac{1}{3}\right)=88}}</math> | ||
|style="text-align:right;"|ואמור י"ב פעמי' ז' ושליש יבואו פ"ח אונקיו' כסף | |style="text-align:right;"|ואמור י"ב פעמי' ז' ושליש יבואו פ"ח אונקיו' כסף | ||
|- | |- | ||
| | | | ||
| − | : | + | :12 times 73 liṭra and 5 dinar are 879 liṭra. |
| + | :<math>\scriptstyle{\color{blue}{12\sdot\left(73+\frac{1}{4}\right)=879}}</math> | ||
|style="text-align:right;"|וי"ב פעמים ע"ג ליטרי' וה' דינרי' יהיו ת"תע"ט ליט‫' | |style="text-align:right;"|וי"ב פעמים ע"ג ליטרי' וה' דינרי' יהיו ת"תע"ט ליט‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | :So, 88 silver ounces are worth 879 liṭra. |
|style="text-align:right;"|הרי שפ"ח אונקיו' כסף שוי' תתע"ט ליטרי‫' | |style="text-align:right;"|הרי שפ"ח אונקיו' כסף שוי' תתע"ט ליטרי‫' | ||
|- | |- | ||
| | | | ||
| − | ::<span style=color: | + | :We want to know how much the 19 ounces are worth. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואנו רוצי' לידע כמה שוי' הי"ט אונקיו' לחשבון זה |
| − | אמור אם כן אם פ"ח אונקיו' כסף שוי' תתע"ט ליטרי' ואנו רוצי' לידע כמה שוי הי"ט אונקיו' לחשבון זה | + | |- |
| + | | | ||
| + | :<span style=color:green>'''Rule of Three:'''</span> Say: if 88 silver ounces are worth 879 liṭra, how much are 19 ounces worth? | ||
| + | :<math>\scriptstyle{\color{blue}{88:879=19:X}}</math> | ||
| + | |style="text-align:right;"|אמור אם כן אם פ"ח אונקיו' כסף שוי' תתע"ט ליטרי' ואנו רוצי' לידע כמה שוי הי"ט אונקיו' לחשבון זה | ||
| + | |- | ||
| + | | | ||
| + | :Say: 19 times 879 is 16701. | ||
| + | |style="text-align:right;"|אמור י"ט פעמי' תתע"ט יהיו י"ו אלפים ותש"א ליט‫' | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{X=\frac{19\sdot879}{88}=\frac{16701}{88}=\frac{16701}{8\sdot11}=189+\frac{15}{20}+\frac{\frac{8+\frac{2}{11}}{12}}{20}}}</math> | + | :Divide it by 88, i.e. by 8 and by 11; the result is 189 liṭra, 15 dinar, 8 pešiṭim and 2 parts of 11 of one pašuṭ and so the 19 silver ounces are worth. |
| − | + | :<math>\scriptstyle{\color{blue}{X=\frac{19\sdot879}{88}=\frac{16701}{88}=\frac{16701}{8\sdot11}=189+\frac{15}{20}+\frac{\frac{8+\frac{2}{11}}{12}}{20}}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|חלק אותם בפ"ח דהיינו בח' ובי"א יבואו קפ"ט ליטרי' וט"ו דינרי' וח' פשיטי' וב' חלקי' מי"א מהפשוט וכן ישוו הי"ט אונקיו' כסף לפי חשבון זה |
| − | חלק אותם בפ"ח דהיינו בח' ובי"א יבואו קפ"ט ליטרי' וט"ו דינרי' וח' פשיטי' וב' חלקי' מי"א מהפשוט וכן ישוו הי"ט אונקיו' כסף לפי חשבון זה | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | === Divide a Quantity Problems === | + | === <span style=color:green>Divide a Quantity Problems</span> === |
| | | | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:money|645|zI1j}}If you want to divide 5 pešiṭim | + | *{{#annot:money|645|zI1j}}If you want to divide 5 pešiṭim into a third and a quarter without a remainder |
:<math>\scriptstyle\frac{1}{3}X+\frac{1}{4}X=5</math> | :<math>\scriptstyle\frac{1}{3}X+\frac{1}{4}X=5</math> | ||
|style="text-align:right;"|אם תרצה לחלק ה' פשיטים לשליש ולרביע ולא ישארו מהם כלל{{#annotend:zI1j}} | |style="text-align:right;"|אם תרצה לחלק ה' פשיטים לשליש ולרביע ולא ישארו מהם כלל{{#annotend:zI1j}} | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{3\sdot4=12}}</math> | + | :<span style=color:green>'''False Position - denominator:'''</span> Say: a third and a quarter are found in 3 times 4, which is 12. |
| + | :<math>\scriptstyle{\color{blue}{3\sdot4=12}}</math> | ||
|style="text-align:right;"|אמור שליש ורביע ימצא בג' פעמים ד' שהם י"ב | |style="text-align:right;"|אמור שליש ורביע ימצא בג' פעמים ד' שהם י"ב | ||
|- | |- | ||
| | | | ||
| − | : | + | :The third is 4; the quarter is 3; their sum is 7 and this is the denominator. |
| − | + | :<math>\scriptstyle{\color{blue}{\left(\frac{1}{3}\sdot12\right)+\left(\frac{1}{4}\sdot12\right)=4+3=7}}</math> | |
|style="text-align:right;"|השליש הוא ד' הרביע הוא ג' וכללם הוא ז' והוא המחלק | |style="text-align:right;"|השליש הוא ד' הרביע הוא ג' וכללם הוא ז' והוא המחלק | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> If you want to know how much is the portion of the one who has a third, say: 4 times 5, since they are 5; the result is 20. Divide them by 7; it is 2 and 6 parts of 7. | |
| − | + | :<math>\scriptstyle{\color{blue}{\frac{1}{3}X=\frac{4\sdot5}{7}=\frac{20}{7}=2+\frac{6}{7}}}</math> | |
|style="text-align:right;"|ואם יאמר תרצה לדעת כמה הוא חלק אותו שיש לו השליש<br> | |style="text-align:right;"|ואם יאמר תרצה לדעת כמה הוא חלק אותו שיש לו השליש<br> | ||
אמור ד' פעמי' ה' בעבור שהם ה' יבואו כ‫'<br> | אמור ד' פעמי' ה' בעבור שהם ה' יבואו כ‫'<br> | ||
| Line 2,877: | Line 3,836: | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> If you want to know the portion of the one who has a quarter, say: 3 times 5 is 15. Divide them by 7; it is 2 and one part of 7. | |
| − | + | :<math>\scriptstyle{\color{blue}{\frac{1}{4}X=\frac{3\sdot5}{7}=\frac{15}{7}=2+\frac{1}{7}}}</math> | |
|style="text-align:right;"|ואם תרצה לדעת חלק אותו שיש לו הרביע<br> | |style="text-align:right;"|ואם תרצה לדעת חלק אותו שיש לו הרביע<br> | ||
אמור ג' פעמי' ה' ט"ו<br> | אמור ג' פעמי' ה' ט"ו<br> | ||
| Line 2,884: | Line 3,843: | ||
|- | |- | ||
| | | | ||
| − | :Check: <math>\scriptstyle{\color{blue}{\left(2+\frac{6}{7}\right)+\left(2+\frac{1}{7}\right)=5}}</math> | + | :<span style=color:green>'''Check:'''</span> If you sum 2 and 6 parts of 7 with 2 and one part of 7; they are 5 pešiṭim, as the amount of money you divided. |
| − | |style="text-align:right;"|ואם תקבץ ב' וו' חלקים מז' וב' וא' חלק מז' יהיו ה' פשיטי' | + | :<math>\scriptstyle{\color{blue}{\left(2+\frac{6}{7}\right)+\left(2+\frac{1}{7}\right)=5}}</math> |
| + | |style="text-align:right;"|ואם תקבץ ב' וו' חלקים מז' וב' וא' חלק מז' יהיו ה' פשיטי' כמספר המעות שחלקת | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:money|645|YiQF}}If you want to divide 12 pešiṭim | + | *{{#annot:money|645|YiQF}}If you want to divide 12 pešiṭim into a half, a third, and a quarter |
:<math>\scriptstyle\frac{1}{2}X+\frac{1}{3}X+\frac{1}{4}X=12</math> | :<math>\scriptstyle\frac{1}{2}X+\frac{1}{3}X+\frac{1}{4}X=12</math> | ||
|style="text-align:right;"|ואם תרצה לחלק י"ב פשי' לחצי לשליש ולרביע{{#annotend:YiQF}} | |style="text-align:right;"|ואם תרצה לחלק י"ב פשי' לחצי לשליש ולרביע{{#annotend:YiQF}} | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''False Position - denominator:'''</span> Say: a half, a third and a quarter are found in 12. | |
| − | ::<math>\scriptstyle{\color{blue}{\left(\frac{1}{2}\sdot12\right)+\left(\frac{1}{3}\sdot12\right)+\left(\frac{1}{4}\sdot12\right)=6+4+3=13}}</math> | + | |style="text-align:right;"|אמור חצי ושליש ורביע ימצאו בי"ב |
| − | + | |- | |
| − | |style="text-align:right;"| | + | | |
| − | החצי הוא ו' השליש הוא ד' הרביע ג' וכללם י"ג והוא המחלק | + | :The half is 6; the third is 4; the quarter is 3; their sum is 13 and this is the denominator. |
| + | :<math>\scriptstyle{\color{blue}{\left(\frac{1}{2}\sdot12\right)+\left(\frac{1}{3}\sdot12\right)+\left(\frac{1}{4}\sdot12\right)=6+4+3=13}}</math> | ||
| + | |style="text-align:right;"|החצי הוא ו' השליש הוא ד' הרביע ג' וכללם י"ג והוא המחלק | ||
| + | |- | ||
| + | | | ||
| + | :If you want to know the share of the one who has a half: | ||
| + | |style="text-align:right;"|ואם תרצה לדעת חלק מי שיש לו החצי | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> Say: a half of 12 is 6. Multiply it by 12, which is the amount of money; it is 72. Divide it by 13; it is 5 and 7 parts of 13. | |
| − | + | :<math>\scriptstyle{\color{blue}{\frac{1}{2}X=\frac{\left(\frac{1}{2}\sdot12\right)\sdot12}{13}=\frac{6\sdot12}{13}=\frac{72}{13}=5+\frac{7}{13}}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אמור החצי מי"ב הוא ו' תרבה אותם עם י"ב שהוא סך המעות יהיו ע"ב<br> |
| − | אמור החצי מי"ב הוא ו' תרבה אותם עם י"ב שהוא סך המעות יהיו ע"ב<br> | ||
חלקם בי"ג יהיו ה' וז' חלקים מי"ג | חלקם בי"ג יהיו ה' וז' חלקים מי"ג | ||
|- | |- | ||
| | | | ||
| − | : | + | :If you want to know the share of the one who has a third: |
| − | + | |style="text-align:right;"|ואם תרצה לדעת חלק אותו שיש לו השליש | |
| − | |style="text-align:right;"| | + | |- |
| − | אמור השליש מי"ב הוא ד' ותרבה אותם עם סך המעות שהם י"ב ויהיו מ"ח<br> | + | | |
| + | :<span style=color:green>'''Rule of Three:'''</span> Say: a third of 12 is 4. Multiply it by the amount of money, which is 12; it is 48. Divide it by 13; it is 3 and 9 parts of 13. | ||
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{3}X=\frac{\left(\frac{1}{3}\sdot12\right)\sdot12}{13}=\frac{4\sdot12}{13}=\frac{48}{13}=3+\frac{9}{13}}}</math> | ||
| + | |style="text-align:right;"|אמור השליש מי"ב הוא ד' ותרבה אותם עם סך המעות שהם י"ב ויהיו מ"ח<br> | ||
וחלקם בי"ג יהיו ג' וט' חלקים מי"ג | וחלקם בי"ג יהיו ג' וט' חלקים מי"ג | ||
|- | |- | ||
| | | | ||
| − | : | + | :If you want to know the share of the one who has a quarter: |
| − | + | |style="text-align:right;"|ואם תרצה לדעת חלק אותו שיש לו הרביע | |
| − | |style="text-align:right;"| | + | |- |
| − | אמור הרביע מי"ב הוא ג' תרבה אותו עם סך המעות שהם י"ב ויהיו ל"ו<br> | + | | |
| + | :<span style=color:green>'''Rule of Three:'''</span> Say: a quarter of 12 is 3. Multiply it by the amount of money, which is 12; it is 36. Divide it by 13; it is 2 and 10 parts of 13. | ||
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{4}X=\frac{\left(\frac{1}{4}\sdot12\right)\sdot12}{13}=\frac{3\sdot12}{13}=\frac{36}{13}=2+\frac{10}{13}}}</math> | ||
| + | |style="text-align:right;"|אמור הרביע מי"ב הוא ג' תרבה אותו עם סך המעות שהם י"ב ויהיו ל"ו<br> | ||
חלקם בי"ג יהיו ב' וי' חלקי' מי"ג | חלקם בי"ג יהיו ב' וי' חלקי' מי"ג | ||
|- | |- | ||
| | | | ||
| − | :Check: <math>\scriptstyle{\color{blue}{\left(5+\frac{7}{13}\right)+\left(3+\frac{9}{13}\right)+\left(2+\frac{10}{13}\right)=12}}</math> | + | :<span style=color:green>'''Check:'''</span> If you sum up these three portions, they are 12, as the amount of money you divided. |
| − | |style="text-align:right;"|ואם תקבץ ג' החלקים האלה יהיו י"ב | + | :<math>\scriptstyle{\color{blue}{\left(5+\frac{7}{13}\right)+\left(3+\frac{9}{13}\right)+\left(2+\frac{10}{13}\right)=12}}</math> |
| + | |style="text-align:right;"|ואם תקבץ ג' החלקים האלה יהיו י"ב כמספר המעו' שחלקת | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:money|645|r4MN}}If you want to divide 12 pešiṭim | + | *{{#annot:money|645|r4MN}}If you want to divide 12 pešiṭim into a third and a quarter |
:<math>\scriptstyle\frac{1}{3}X+\frac{1}{4}X=12</math> | :<math>\scriptstyle\frac{1}{3}X+\frac{1}{4}X=12</math> | ||
|style="text-align:right;"|וכן אם תרצה לחלק י"ב פשיטי' לשליש ולרביע{{#annotend:r4MN}} | |style="text-align:right;"|וכן אם תרצה לחלק י"ב פשיטי' לשליש ולרביע{{#annotend:r4MN}} | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''False Position - denominator:'''</span> Say: a third and a quarter are found in 12. | |
| − | |style="text-align:right;"|אמור שליש ורבי' ימצאו א בי"ב | + | |style="text-align:right;"|אמור שליש ורבי' ימצאו א בי"ב |
|- | |- | ||
| | | | ||
| − | : | + | :The third is 4; the quarter is 3; their sum is 7 and this is the denominator. |
| − | + | :<math>\scriptstyle{\color{blue}{\left(\frac{1}{3}\sdot12\right)+\left(\frac{1}{4}\sdot12\right)=4+3=7}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|השליש הוא ד' והרביע הוא ג' וכללם ז' והוא המחלק |
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :If you want to know how much is [the share of] the one who has a third: |
| − | + | |style="text-align:right;"|ואם תרצה לדעת מי שיש לו השליש כמה יהיו | |
| − | |style="text-align:right;"| | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | :Check: <math>\scriptstyle{\color{blue}{\left(6+\frac{6}{7}\right)+\left(5+\frac{1}{7}\right)=12}}</math> | + | :<span style=color:green>'''Rule of Three:'''</span> Say: a third of 12 is 4. Multiply it by the amount of money, which is 12; it is 48. Divide it by 7; it is 6 and 6 parts of 7. |
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{3}X=\frac{\left(\frac{1}{3}\sdot12\right)\sdot12}{7}=\frac{4\sdot12}{7}=\frac{48}{7}=6+\frac{6}{7}}}</math> | ||
| + | |style="text-align:right;"|אמור שליש מי"ב הם ד' תרבה אותם על סך המעות שיהיו י"ב ויהיו מ"ח<br> | ||
| + | חלקם בז' יהיו ו' וו' חלקי' מז‫' | ||
| + | |- | ||
| + | | | ||
| + | :To know [how much is the share of] the one who has a quarter: | ||
| + | |style="text-align:right;"|ולדעת אותו שיש לו הרביע | ||
| + | |- | ||
| + | | | ||
| + | :<span style=color:green>'''Rule of Three:'''</span> Say: a quarter of 12 is 3. Multiply it by 12; the quarter is 36. Divide it by 7; it is 5 and 1 part of 7. | ||
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{4}X=\frac{\left(\frac{1}{4}\sdot12\right)\sdot12}{7}=\frac{3\sdot12}{7}=\frac{36}{7}=5+\frac{1}{7}}}</math> | ||
| + | |style="text-align:right;"|אמור הרביע מי"ב הוא ג' תרבה ‫<ref>38r</ref>אותם עם י"ב יהיה הרביע ל"ו<br> | ||
| + | חלקם בז' יהיו ה' וא' חלק מז‫' | ||
| + | |- | ||
| + | | | ||
| + | :<span style=color:green>'''Check:'''</span> The [sum of the] two is 12. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(6+\frac{6}{7}\right)+\left(5+\frac{1}{7}\right)=12}}</math> | ||
|style="text-align:right;"|ושניהם י"ב | |style="text-align:right;"|ושניהם י"ב | ||
|- | |- | ||
| Line 2,954: | Line 3,938: | ||
*{{#annot:money|645|LSBW}}If you have 30 pešiṭim and you want to divide them to a third, a quarter, a fifth, and a sixth | *{{#annot:money|645|LSBW}}If you have 30 pešiṭim and you want to divide them to a third, a quarter, a fifth, and a sixth | ||
:<math>\scriptstyle\frac{1}{3}X+\frac{1}{4}X+\frac{1}{5}X+\frac{1}{6}X=30</math> | :<math>\scriptstyle\frac{1}{3}X+\frac{1}{4}X+\frac{1}{5}X+\frac{1}{6}X=30</math> | ||
| − | |style="text-align:right;"|ואם יהיו בידך ל' פשיטי' ותרצה לחלק אותם לשליש ולרביע ולחומש ולשתות{{#annotend:LSBW}} | + | |style="text-align:right;"|<big>ואם יהיו</big> בידך ל' פשיטי' ותרצה לחלק אותם לשליש ולרביע ולחומש ולשתות{{#annotend:LSBW}} |
| + | |- | ||
| + | | | ||
| + | :<span style=color:green>'''False Position - denominator:'''</span> Say: these fractions are found in 60. | ||
| + | |style="text-align:right;"|אמור החלקי' האלה ימצאו בס‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | :The third is 20; the quarter is 15; the fifth is 12; the sixth is 10; their sum is 57 and this is the denominator. |
| − | + | |style="text-align:right;"|השליש הוא כ' הרביע ט"ו החומש י"ב השתות י' וכלם נ"ז והוא המחלק | |
| − | + | |- | |
| − | + | | colspan="2"| | |
| + | :<math>\scriptstyle{\color{blue}{\left(\frac{1}{3}\sdot60\right)+\left(\frac{1}{4}\sdot60\right)+\left(\frac{1}{5}\sdot60\right)+\left(\frac{1}{6}\sdot60\right)=20+15+12+10=57}}</math> | ||
|- | |- | ||
| | | | ||
| − | : | + | : |
| − | + | :<span style=color:green>'''Rule of Three:'''</span> For the one who has a third, say: a third of 60 is 20. Multiply it by 30, which is the amount of money; it is 600. Divide it by 57; the result is 10 and 30 parts of 57. | |
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{3}X=\frac{\left(\frac{1}{3}\sdot60\right)\sdot30}{57}=\frac{20\sdot30}{57}=\frac{600}{57}=10+\frac{30}{57}}}</math> | ||
|style="text-align:right;"|ואותו שיש לו השליש יאמר השליש מס' הוא כ' ותרבה אותם עם ל' שהוא סך המעות יהיו ת"ר<br> | |style="text-align:right;"|ואותו שיש לו השליש יאמר השליש מס' הוא כ' ותרבה אותם עם ל' שהוא סך המעות יהיו ת"ר<br> | ||
חלקי' בנ"ז יבואו י' ול' חלקי' מנ"ז | חלקי' בנ"ז יבואו י' ול' חלקי' מנ"ז | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> For the one who has a quarter, which is 15, say: 15 times 30, is 450. Divide it by 57; it is 7 and 51 parts of 57. | |
| − | + | :<math>\scriptstyle{\color{blue}{\frac{1}{4}X=\frac{\left(\frac{1}{4}\sdot60\right)\sdot30}{57}=\frac{15\sdot30}{57}=\frac{450}{57}=7+\frac{51}{57}}}</math> | |
|style="text-align:right;"|ואותו שיש לו הרביע שהוא ט"ו יאמר ט"ו פעמי' ל' הם ת"ן<br> | |style="text-align:right;"|ואותו שיש לו הרביע שהוא ט"ו יאמר ט"ו פעמי' ל' הם ת"ן<br> | ||
חלקם בנ"ז יהיו ז' ונ"א חלקי' מנ"ז | חלקם בנ"ז יהיו ז' ונ"א חלקי' מנ"ז | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> For the one who has a fifth of [60], which is 12, say: 12 times 30, is 360. Divide it by 57; the result is 6 and 18 parts of 57. | |
| − | + | :<math>\scriptstyle{\color{blue}{\frac{1}{5}X=\frac{\left(\frac{1}{5}\sdot60\right)\sdot30}{57}=\frac{12\sdot30}{57}=\frac{360}{57}=6+\frac{18}{57}}}</math> | |
|style="text-align:right;"|ואותו שיש לו החומש מי"ב שהוא י"ב יאמר י"ב פעמי' ל' הם ש"ס<br> | |style="text-align:right;"|ואותו שיש לו החומש מי"ב שהוא י"ב יאמר י"ב פעמי' ל' הם ש"ס<br> | ||
חלקם בנ"ז יבאו ו' וי"ח חלקי' מנ"ז | חלקם בנ"ז יבאו ו' וי"ח חלקי' מנ"ז | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> For the one who has a sixth, which is 10, say: 10 times 30, is 300. Divide it by 57; it is 5 and 15 parts of 57. | |
| − | + | :<math>\scriptstyle{\color{blue}{\frac{1}{6}X=\frac{\left(\frac{1}{6}\sdot60\right)\sdot30}{57}=\frac{10\sdot30}{57}=\frac{300}{57}=5+\frac{15}{57}}}</math> | |
| − | |style="text-align:right;"|ואותו שיש לו השתות שהוא י' יאמר י' פעמי' ל' הם ש‫'<br> | + | |style="text-align:right;"|ואותו שיש לו השתות שהוא י' יאמר י' פעמי' ל' הם ש"ס ש‫'<br> |
חלקם בנ"ז יהיו ה' וט"ו חלקי' מנ"ז | חלקם בנ"ז יהיו ה' וט"ו חלקי' מנ"ז | ||
|- | |- | ||
| | | | ||
| − | :Check: <math>\scriptstyle{\color{blue}{\left(10+\frac{30}{57}\right)+\left(7+\frac{51}{57}\right)+\left(6+\frac{18}{57}\right)+\left(5+\frac{15}{57}\right)=30}}</math> | + | :<span style=color:green>'''Check:'''</span> The total sum of all the mentioned portions is 30 pešiṭim. |
| + | :<math>\scriptstyle{\color{blue}{\left(10+\frac{30}{57}\right)+\left(7+\frac{51}{57}\right)+\left(6+\frac{18}{57}\right)+\left(5+\frac{15}{57}\right)=30}}</math> | ||
|style="text-align:right;"|וכלל כל החלקי' הנזכרי' הם ל' פשיטי‫' | |style="text-align:right;"|וכלל כל החלקי' הנזכרי' הם ל' פשיטי‫' | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:money|645|fRzF}}If you want to divide 60 liṭra | + | *{{#annot:money|645|fRzF}}If you want to divide 60 liṭra into a half, a third, and a quarter |
:<math>\scriptstyle\frac{1}{2}X+\frac{1}{3}X+\frac{1}{4}X=60</math> | :<math>\scriptstyle\frac{1}{2}X+\frac{1}{3}X+\frac{1}{4}X=60</math> | ||
|style="text-align:right;"|לחלק ס' ליטרי' לחצי לשליש ולרביע{{#annotend:fRzF}} | |style="text-align:right;"|לחלק ס' ליטרי' לחצי לשליש ולרביע{{#annotend:fRzF}} | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''False Position - denominator:'''</span> Say: a half, a third and a quarter are found in 12. | |
| − | |style="text-align:right;"|אמור חצי ושליש ורביע ימצאו בי"ב | + | |style="text-align:right;"|אמור חצי ושליש ורביע ימצאו בי"ב |
|- | |- | ||
| | | | ||
| − | : | + | :Their sum is 13 and this is the denominator. |
| − | + | :<math>\scriptstyle{\color{blue}{\left(\frac{1}{2}\sdot12\right)+\left(\frac{1}{3}\sdot12\right)+\left(\frac{1}{4}\sdot12\right)=13}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכללם י"ג והוא המחלק |
| − | אמור החצי מי"ב הוא ו' תרבה אות' עם ס' שהוא סכום ה' ליטרי' יהיו ש"ס<br> | + | |- |
| + | | | ||
| + | :If you want to know the share of the one who has a half: | ||
| + | |style="text-align:right;"|ואם תרצה לדעת חלק אותו שיש לו החצי | ||
| + | |- | ||
| + | | | ||
| + | :<span style=color:green>'''Rule of Three:'''</span> Say: a half of 12 is 6. Multiply it by 60, which is the number of liṭra; the result is 360. Divide them by 13; it is 27 and 9 parts of 13 of one liṭra. | ||
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{2}X=\frac{\left(\frac{1}{2}\sdot12\right)\sdot60}{13}=\frac{6\sdot60}{13}=\frac{360}{13}=27+\frac{9}{13}}}</math> | ||
| + | |style="text-align:right;"|אמור החצי מי"ב הוא ו' תרבה אות' עם ס' שהוא סכום ה' ליטרי' יהיו ש"ס<br> | ||
חלקם בי"ג יהיו כ"ז וט' חלקים מי"ג מהליטר‫' | חלקם בי"ג יהיו כ"ז וט' חלקים מי"ג מהליטר‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | :If you want to know the share of the one who has a third: |
| − | + | |style="text-align:right;"|ואם תרצה לדעת חלק אותו שיש לו השליש | |
| − | |style="text-align:right;"| | + | |- |
| − | אמור השליש מי"ב הם ד' תרבה אותם עם ס' יהיו ר"מ<br> | + | | |
| − | חלקם בי"ג יהיו י"ח וו' חלקי' מי"ג מן הליטר‫' | + | :<span style=color:green>'''Rule of Three:'''</span> Say: a third of 12 is 4. Multiply it by 60; it is 240. Divide them by 13; it is 18 and 6 parts of 13 of one liṭra. |
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{3}X=\frac{\left(\frac{1}{3}\sdot12\right)\sdot60}{13}=\frac{4\sdot60}{13}=\frac{240}{13}=18+\frac{6}{13}}}</math> | ||
| + | |style="text-align:right;"|אמור השליש מי"ב הם ד' תרבה אותם עם ס' יהיו ר"מ<br> | ||
| + | חלקם בי"ג יהיו יהיו י"ח וו' חלקי' מי"ג מן הליטר‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | :If you want to know the share of the one who has a quarter: |
| − | + | |style="text-align:right;"|ואם תרצה לדעת אותו שיש לו הרביע | |
| − | |style="text-align:right;"| | + | |- |
| − | אמור הרביע מי"ב הוא ג' תרבה אותם עם ס' יהיו ק"פ<br> | + | | |
| + | :<span style=color:green>'''Rule of Three:'''</span> Say: a quarter of 12 is 3. Multiply it by 60; it is 180. Divide them by 13; it is 13 and 11 parts of 13 of one liṭra. | ||
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{4}X=\frac{\left(\frac{1}{4}\sdot12\right)\sdot60}{13}=\frac{3\sdot60}{13}=\frac{180}{13}=13+\frac{11}{13}}}</math> | ||
| + | |style="text-align:right;"|אמור הרביע מי"ב הוא ג' תרבה אותם עם ס' יהיו ק"פ<br> | ||
חלקם בי"ג הוא י"ג וי"א חלקי' מי"ג מן הליט‫' | חלקם בי"ג הוא י"ג וי"א חלקי' מי"ג מן הליט‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | :1 part of 13 of one liṭra is 18 pešiṭim and [6] parts of 13. |
| − | + | :<math>\scriptstyle{\color{blue}{\frac{1}{13}=\frac{18+\frac{6}{13}}{20\sdot12}}}</math> | |
| − | |style="text-align:right;"|וא' חלק מי"ג מן הליטר' הוא י"ח פשיטי' | + | |style="text-align:right;"|וא' חלק מי"ג מן הליטר' הוא י"ח פשיטי' וב' חלקי' מי"ג |
|- | |- | ||
| | | | ||
| − | : | + | :So, the share of the one who has a half is 27 liṭra, 13 dinar, 10 pešiṭim and 2 parts of 13 of one pašuṭ. |
| − | + | :<math>\scriptstyle{\color{blue}{\frac{1}{2}X=27+\frac{9}{13}=27+\frac{13}{20}+\frac{10+\frac{2}{13}}{20\sdot12}}}</math> | |
| − | |||
|style="text-align:right;"|יהיה אם כן חלק אותו שיש לו החצי כ"ז ליטרי' וי"ג דינרי' וי' פשיטי' וב' חלקי' מי"ג מן הפשוט | |style="text-align:right;"|יהיה אם כן חלק אותו שיש לו החצי כ"ז ליטרי' וי"ג דינרי' וי' פשיטי' וב' חלקי' מי"ג מן הפשוט | ||
|- | |- | ||
| | | | ||
| − | : | + | :The share of the one who has a third is 18 liṭra, 9 dinar, 2 pešiṭim and 10 parts of 13 of one pašuṭ. |
| − | + | :<math>\scriptstyle{\color{blue}{\frac{1}{3}X=18+\frac{6}{13}=18+\frac{9}{20}+\frac{2+\frac{10}{13}}{20\sdot12}}}</math> | |
| − | |||
|style="text-align:right;"|וחלק אותו שיש לו השליש הוא י"ח ליטרי' וט' דינרי' וב' פשיטי' וי' חלקי' מי"ג מן הפשוט | |style="text-align:right;"|וחלק אותו שיש לו השליש הוא י"ח ליטרי' וט' דינרי' וב' פשיטי' וי' חלקי' מי"ג מן הפשוט | ||
|- | |- | ||
| | | | ||
| − | : | + | :The share of the one who has a quarter is 13 liṭra, 16 dinar, 11 pešiṭim and 1 part of 13 of one pašuṭ. |
| − | + | :<math>\scriptstyle{\color{blue}{\frac{1}{4}X=13+\frac{11}{13}=13+\frac{16}{20}+\frac{11+\frac{1}{13}}{20\sdot12}}}</math> | |
| − | |||
|style="text-align:right;"|וחלק אותו שיש לו הרביע הוא י"ג ליטרי' וי"ו דינרי' וי"א פשיטי' וא' חלק מי"ג מן הפשוט | |style="text-align:right;"|וחלק אותו שיש לו הרביע הוא י"ג ליטרי' וי"ו דינרי' וי"א פשיטי' וא' חלק מי"ג מן הפשוט | ||
|- | |- | ||
| | | | ||
| − | :Check: | + | :<span style=color:green>'''Check:'''</span> If you sum up all the mentioned portions, they are 60 liṭra. |
| + | |style="text-align:right;"|ואם תקבץ כל החלקים הנזכרי' יהיו ס' ליטרי‫' | ||
| + | |- | ||
| + | | colspan="2"| | ||
:<math>\scriptstyle{\color{blue}{\left(27+\frac{13}{20}+\frac{10+\frac{2}{13}}{20\sdot12}\right)+\left(18+\frac{9}{20}+\frac{2+\frac{10}{13}}{20\sdot12}\right)+\left(13+\frac{16}{20}+\frac{11+\frac{1}{13}}{20\sdot12}\right)=60}}</math> | :<math>\scriptstyle{\color{blue}{\left(27+\frac{13}{20}+\frac{10+\frac{2}{13}}{20\sdot12}\right)+\left(18+\frac{9}{20}+\frac{2+\frac{10}{13}}{20\sdot12}\right)+\left(13+\frac{16}{20}+\frac{11+\frac{1}{13}}{20\sdot12}\right)=60}}</math> | ||
| − | |||
|- | |- | ||
| | | | ||
| − | === Partnership - for the same time === | + | === <span style=color:green>Partnership - for the same time</span> === |
| | | | ||
| Line 3,061: | Line 4,065: | ||
:How much gold should each of them contribute so that the total will be 40 ounces and the share of each of them will be equal to the shares of his friends?<br> | :How much gold should each of them contribute so that the total will be 40 ounces and the share of each of them will be equal to the shares of his friends?<br> | ||
:<math>\scriptstyle\frac{1}{3}X+\frac{1}{5}X+\frac{1}{8}X=40</math> | :<math>\scriptstyle\frac{1}{3}X+\frac{1}{5}X+\frac{1}{8}X=40</math> | ||
| − | |style="text-align:right;"|שלשה אנשים עשו חברותה ושמו בין שלשתם א' אונקי' זהב<br> | + | |style="text-align:right;"|<big>שלשה אנשים עשו חברותה ושמו בין שלשתם</big> א' אונקי' זהב<br> |
לאחד היה זהב שוה ג' ליטרי' האונקי‫'<br> | לאחד היה זהב שוה ג' ליטרי' האונקי‫'<br> | ||
ולשני זהב שוה ה' ליטרי' האונקי‫'<br> | ולשני זהב שוה ה' ליטרי' האונקי‫'<br> | ||
| Line 3,068: | Line 4,072: | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''False Position - denominator:'''</span> Say: a third, a fifth, and an eighth are found is 120. | |
| − | |style="text-align:right;"|אמור שליש וחומש ושמין ימצאו בק"כ | + | :<math>\scriptstyle{\color{blue}{\left(\frac{1}{3}\sdot120\right)+\left(\frac{1}{5}\sdot120\right)+\left(\frac{1}{8}\sdot120\right)=40+24+15=79}}</math> |
| − | השליש הוא מ' החומש הוא כ"ד השמינית ט"ו וכללם ע"ט והוא המחלק | + | |style="text-align:right;"|אמור שליש וחומש ושמין ימצאו בק"כ |
| + | |- | ||
| + | | | ||
| + | :The third is 40; the fifth is 24; the eighth is 15; their total sum is 79 and this is the denominator. | ||
| + | |style="text-align:right;"|השליש הוא מ' החומש הוא כ"ד השמינית ט"ו וכללם ע"ט והוא המחלק | ||
|- | |- | ||
| | | | ||
| − | : | + | :If you want to know how much gold the one whose gold is worth 3 liṭra for an ounce contributes, say: the third of 120 is 40. Multiply it by the number of ounces, which is 40; it is 1600. Divide it by 79; the result is 20 ounces and 20 parts of 79. |
| − | + | :<math>\scriptstyle{\color{blue}{\frac{1}{3}X=\frac{\left(\frac{1}{3}\sdot120\right)\sdot40}{79}=\frac{40\sdot40}{79}=\frac{1600}{79}=20+\frac{20}{79}}}</math> | |
| − | |style="text-align:right;"|ואם תרצה לדעת כמה זהב ישים אותו שהזהב שלו שוה ג' ליטרי' האונקי‫'<br> | + | |style="text-align:right;"|<big>ואם תרצה</big> לדעת כמה זהב ישים אותו שהזהב שלו שוה ג' ליטרי' האונקי‫'<br> |
| − | אמור השליש מק"כ הוא מ' ותרבה אותו עם סך האונקיות שהם מ' יהיו אלף ות"ר<br> | + | אמור השליש מק"כ הוא מ' ותרבה אותו עם סך האונקיות ‫<ref>38v</ref>שהם מ' יהיו אלף ות"ר‫<ref>לדעת כמה זהב ישים... יהיו אלף ות"ר: MS ithaca twice</ref><br> |
חלקם בע"ט יביאו כ' אונקיו' זהב וכ' חלקי' מע"ט | חלקם בע"ט יביאו כ' אונקיו' זהב וכ' חלקי' מע"ט | ||
|- | |- | ||
| | | | ||
| − | :::<math>\scriptstyle{\color{blue}{3\sdot20=60}}</math> | + | :If you want to know how much his share is worth, say: the 20 ounces at 3 liṭra for an ounce are worth 60 liṭra. |
| − | |style="text-align:right;"|ואם תרצה לדעת כמה שוה חלקו אמור הכ' אונקיו' לחשבון ג' | + | :<math>\scriptstyle{\color{blue}{3\sdot20=60}}</math> |
| + | |style="text-align:right;"|<big>ואם תרצה</big> לדעת כמה שוה חלקו אמור הכ' אונקיו' לחשבון ג' לי' האונ' עולי' ס' ליטרי‫' | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> for the 20 parts of 79 say: if 79, which is 1 ounce, is worth 720 pešuṭim that are 3 liṭra, how much are 20 parts of 79 worth? | |
| − | |style="text-align:right;"|ומן הכ' חלקי' מע"ט אמור אם ע"ט שהוא כ' ליטרי' א' | + | :<math>\scriptstyle{\color{blue}{1:720=\frac{20}{79}:X}}</math> |
| + | |style="text-align:right;"|ומן הכ' חלקי' מע"ט אמור אם ע"ט שהוא כ' ליטרי' א' אונקי' שוה תש"כ פשוטי' שהם ג' ליט' כ' חלקים מע"ט כמה שווים | ||
|- | |- | ||
| | | | ||
| − | :::<math>\scriptstyle{\color{blue}{\frac{720\sdot20}{79}=\frac{14400}{79}=182+\frac{22}{79}}}</math> | + | :Say: 20 times 720 is 14400. Divide it by 79; the result is 182 pešiṭim and 22 parts of 79. |
| − | |style="text-align:right;"|אמו' כ' פעמ' תש"כ | + | :<math>\scriptstyle{\color{blue}{\frac{720\sdot20}{79}=\frac{14400}{79}=182+\frac{22}{79}}}</math> |
| + | |style="text-align:right;"|אמו' כ' פעמ' תש"כ‫<ref>פשוטי' שהם ג'... תש"כ: MS Ithaca om.</ref> יהיו <s>ד</s> י"ד אלפי' ות‫'<br> | ||
חלקם בע"ט יבואו קפ"ב פשיטי' וכ"ב חלקים מע"ט | חלקם בע"ט יבואו קפ"ב פשיטי' וכ"ב חלקים מע"ט | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{X=60+\frac{182+\frac{22}{79}}{20\sdot12}=60+\frac{15}{20}+\frac{2+\frac{22}{79}}{20\sdot12}}}</math> | + | :Which are [60 liṭra], 15 dinar, 2 pešuṭim and 22 parts of 79. |
| − | + | :<math>\scriptstyle{\color{blue}{X=60+\frac{182+\frac{22}{79}}{20\sdot12}=60+\frac{15}{20}+\frac{2+\frac{22}{79}}{20\sdot12}}}</math> | |
| − | |style="text-align:right;"|שהם ס' ליט' וט"ו דינרי' וב"פ וכ"ב חלקים מע"ט | + | |style="text-align:right;"|שהם ס' ליט'‫<ref>ס' ליט': MS Ithaca כ"ה אלפים</ref> וט"ו דינרי' וב"פ וכ"ב חלקים מע"ט |
|- | |- | ||
| | | | ||
| − | : | + | :If you want to know how much gold the one whose gold is worth 5 liṭra for an ounce contributes, say: the fifth of 120 is 24. Multiply it by 40, which is the number of ounces; the result is 960. Divide it by 79; [it is] 12 ounces and 12 parts of 79. |
| − | + | :<math>\scriptstyle{\color{blue}{\frac{1}{5}X=\frac{\left(\frac{1}{5}\sdot120\right)\sdot40}{79}=\frac{24\sdot40}{79}=\frac{960}{79}=12+\frac{12}{79}}}</math> | |
| − | |style="text-align:right;"|ואם תרצה לדעת כמה זהב ישים אותו שהזהב שלו שוה ה' ליטרי' האונ‫'<br> | + | |style="text-align:right;"|<big>ואם תרצה לדעת</big> כמה זהב ישים אותו שהזהב שלו שוה ה' ליטרי' האונ‫'<br> |
| − | אמור החומש מק | + | אמור החומש <s>מק"פ</s> מק"כ‫<ref>מק"כ: MS Ithaca marg.</ref> הם כ"ד תרבה אותם עם מ' שהוא סכום האונקי' עולים תתק"ס<br> |
חלקם בע"ט י"ב אונקיו' וי"ב חלקי' מע"ט | חלקם בע"ט י"ב אונקיו' וי"ב חלקי' מע"ט | ||
|- | |- | ||
| | | | ||
| − | :::<math>\scriptstyle{\color{blue}{5\sdot12=60}}</math> | + | :If you want to know how much his share is worth, say: the 12 ounces at 5 liṭra for an ounce are worth 60 liṭra. |
| − | |style="text-align:right;"|ואם תרצה לדעת כמה שוה חלקו אמור הי"ב אונקיו' לחשבון ה' ליטרי' האונקי' עולי' ס' ליטרי‫' | + | :<math>\scriptstyle{\color{blue}{5\sdot12=60}}</math> |
| + | |style="text-align:right;"|<big>ואם תרצה</big> לדעת כמה שוה חלקו אמור הי"ב אונקיו' לחשבון ה' ליטרי' האונקי' עולי' ס' ליטרי‫' | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> for the 12 parts of 79 say: if 79, which is 1 ounce, is worth 1200 pešuṭim that are 5 liṭra, how much are 12 parts of 79 worth? | |
| − | |style="text-align:right;"|ומן הי"ב חלקים מע"ט אמור אם ע"ט שהוא א' אונקי' עולה אלף ור' פשוטי' שהם ה' ליט' הי"ב חלקים מע"ט כמה שווים | + | :<math>\scriptstyle{\color{blue}{1:1200=\frac{12}{79}:X}}</math> |
| + | |style="text-align:right;"|ומן הי"ב חלקים‫<ref>חלקים: MS Ithaca ליטרי'</ref> מע"ט [אמור אם ע"ט שהוא א' אונקי' עולה אלף ור' פשוטי' שהם ה' ליט' הי"ב חלקים מע"ט כמה שווים | ||
|- | |- | ||
| | | | ||
| − | :::<math>\scriptstyle{\color{blue}{\frac{1200\sdot12}{79}=\frac{14400}{79}=182+\frac{22}{79}}}</math> | + | :Say: 12 times 120 is 14400. Divide it by 79; the result is 182 pešiṭim and 22 parts of 79. |
| + | :<math>\scriptstyle{\color{blue}{\frac{1200\sdot12}{79}=\frac{14400}{79}=182+\frac{22}{79}}}</math> | ||
|style="text-align:right;"|אמו' י"ב פעמ' אלף ור' הם י"ד אלפים ות‫'<br> | |style="text-align:right;"|אמו' י"ב פעמ' אלף ור' הם י"ד אלפים ות‫'<br> | ||
חלקם בע"ט יבואו קפ"ב פשוטי' וכ"ב חלקים מע"ט | חלקם בע"ט יבואו קפ"ב פשוטי' וכ"ב חלקים מע"ט | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{X=60+\frac{182+\frac{22}{79}}{20\sdot12}=60+\frac{15}{20}+\frac{2+\frac{22}{79}}{20\sdot12}}}</math> | + | :So, his share is worth 60 liṭra, 15 dinar, 2 pešiṭim and 22 parts of 79, as the first. |
| − | + | :<math>\scriptstyle{\color{blue}{X=60+\frac{182+\frac{22}{79}}{20\sdot12}=60+\frac{15}{20}+\frac{2+\frac{22}{79}}{20\sdot12}}}</math> | |
|style="text-align:right;"|הרי ששווה חלקו ס' ליט' וט"ו די' וב"פ וכ"ב חלקים מע"ט כמו הראשון | |style="text-align:right;"|הרי ששווה חלקו ס' ליט' וט"ו די' וב"פ וכ"ב חלקים מע"ט כמו הראשון | ||
|- | |- | ||
| | | | ||
| − | : | + | :If you want to know how much gold the one whose gold is worth 8 liṭra for an ounce contributes, say: the eighth of 120 is 15. Multiply it by 40, which is the number of ounces; it is 600. Divide it by 79; the result is 7 ounces and 47 parts of 79. |
| − | + | :<math>\scriptstyle{\color{blue}{\frac{1}{8}X=\frac{\left(\frac{1}{8}\sdot120\right)\sdot40}{79}=\frac{15\sdot40}{79}=\frac{600}{79}=7+\frac{47}{79}}}</math> | |
|style="text-align:right;"|ואם תרצה לדעת כמה זהב ישים אותו שהזהב שלו שווה ח' ליט' האונק‫'<br> | |style="text-align:right;"|ואם תרצה לדעת כמה זהב ישים אותו שהזהב שלו שווה ח' ליט' האונק‫'<br> | ||
אמו' השמינית מק"כ הם ט"ו תרבה אותם עם מ' שהם סכום האונק' יהיו ת"ר<br> | אמו' השמינית מק"כ הם ט"ו תרבה אותם עם מ' שהם סכום האונק' יהיו ת"ר<br> | ||
| Line 3,130: | Line 4,144: | ||
|- | |- | ||
| | | | ||
| − | :::<math>\scriptstyle{\color{blue}{8\sdot7=56}}</math> | + | :If you want to know how much his share is worth, say: the 7 ounces at 8 liṭra [for an ounce] are worth 56 liṭra. |
| + | :<math>\scriptstyle{\color{blue}{8\sdot7=56}}</math> | ||
|style="text-align:right;"|ואם תרצה לדעת כמה שוה חלקו שלזה אמו' הז' אונק' לחשבו' ח' ליט' עולים נ"ו ליט‫' | |style="text-align:right;"|ואם תרצה לדעת כמה שוה חלקו שלזה אמו' הז' אונק' לחשבו' ח' ליט' עולים נ"ו ליט‫' | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> for the 47 parts of 79 say: if 79, which is 1 ounce, is worth 1920 pešuṭim that are 8 liṭra, how much are 47 parts of 79 worth? | |
| + | :<math>\scriptstyle{\color{blue}{1:1920=\frac{47}{79}:X}}</math> | ||
|style="text-align:right;"|ומן המ"ז חלקים מע"ט אמו' אם ע"ט שהוא א' אונק' שוה אלף ותתק"כ פשוט' שהוא ח' ליט' המ"ז חלקים מע"ט כמה שווים | |style="text-align:right;"|ומן המ"ז חלקים מע"ט אמו' אם ע"ט שהוא א' אונק' שוה אלף ותתק"כ פשוט' שהוא ח' ליט' המ"ז חלקים מע"ט כמה שווים | ||
|- | |- | ||
| | | | ||
| − | :::<math>\scriptstyle{\color{blue}{\frac{1920\sdot47}{79}=\frac{90240}{79}=1142+\frac{22}{79}}}</math> | + | :Say: 47 times 1920 is 90240. Divide it by 79; it is 1142 pešiṭim and 22 parts of 79. |
| − | + | :<math>\scriptstyle{\color{blue}{\frac{1920\sdot47}{79}=\frac{90240}{79}=1142+\frac{22}{79}}}</math> | |
|style="text-align:right;"|אמו' מ"ז פעמ' אלף ותתק"כ הם צ' אלפים ור"מ<br> | |style="text-align:right;"|אמו' מ"ז פעמ' אלף ותתק"כ הם צ' אלפים ור"מ<br> | ||
| − | חלקם בע"ט יהיו אלף וקמ"ב פשוטי' וכ"ב חלקים מע"ט< | + | חלקם בע"ט יהיו אלף וקמ"ב פשוטי' וכ"ב חלקים מע"ט |
| − | שהם ד' ליט' וט"ו די' וב"פ וכ"ב חלקים מע"ט | + | |- |
| + | | | ||
| + | :Which are 4 liṭra, 15 dinar, 2 pešiṭim and 22 parts of 79. | ||
| + | :<math>\scriptstyle{\color{blue}{4+\frac{15}{20}+\frac{2+\frac{22}{79}}{20\sdot12}}}</math> | ||
| + | |style="text-align:right;"|שהם ד' ליט' וט"ו די' וב"פ וכ"ב חלקים מע"ט | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{X=56+\left(4+\frac{15}{20}+\frac{2+\frac{22}{79}}{20\sdot12}\right)=60+\frac{15}{20}+\frac{2+\frac{22}{79}}{20\sdot12}}}</math> | + | :Add them to the 56 liṭra, so his share is worth the same as each of his friends: 60 liṭra, 15 dinar, 2 pešiṭim and 22 parts of 79. |
| − | + | :<math>\scriptstyle{\color{blue}{X=56+\left(4+\frac{15}{20}+\frac{2+\frac{22}{79}}{20\sdot12}\right)=60+\frac{15}{20}+\frac{2+\frac{22}{79}}{20\sdot12}}}</math> | |
| − | |style="text-align:right;"|צרף אותם עם הנ"ו ליט' הרי ששווה חלקו שלזה כחלק כל אחד מחבריו ס' ליט' וט"ו די' וב"פ וכ"ב חלקים מע"ט | + | |style="text-align:right;"|צרף אותם עם הנ"ו ליט' הרי ששווה חלקו שלזה כחלק כל אחד מחבריו ס' ליט' וט"ו די' וב"פ וכ"ב חלקים מע"ט]‫<ref>MS Ithaca om. שלשתי ארבעי' אונקיו' זהב</ref> |
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | === Multiple Quantities Problems === | + | === <span style=color:green>Multiple Quantities Problems</span> === |
| | | | ||
| Line 3,163: | Line 4,181: | ||
:The sum of what all the three have is 60. | :The sum of what all the three have is 60. | ||
:How much does each of them have? | :How much does each of them have? | ||
| − | + | |style="text-align:right;"|<big>שלשה אחים לשיני יש יותר מן הראשו'</big> על אחת ג‫'<br> | |
| − | |style="text-align:right;"|שלשה אחים | ||
| − | לשיני יש יותר מן הראשו' על אחת ג‫'<br> | ||
ולשלישי יש לאחת על אחת שיני ג‫'<br> | ולשלישי יש לאחת על אחת שיני ג‫'<br> | ||
ומחובר שלשתן ס‫'<br> | ומחובר שלשתן ס‫'<br> | ||
כמה יש לכל אחת מהן{{#annotend:kyw1}} | כמה יש לכל אחת מהן{{#annotend:kyw1}} | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle a_1+a_2+a_3=60\\\scriptstyle a_2=3a_1\\\scriptstyle a_3=3a_2\end{cases}</math> | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''False Position - denominator:'''</span> Find a denominator [of their sum], for instance, let them be 1, 3, 9; the sum of all three is 13; the first has 1, the second has 3, and the third has 9. | |
| + | :<math>\scriptstyle{\color{blue}{1+3+9=13}}</math> | ||
|style="text-align:right;"|עשה לך מורה שיוסיף לך זה על זה כזה הדמיון והם א' ג' ט' ומחובר שלשתם י"ג יהיה לראשון א' ולשני ג' ולשלישי ט‫' | |style="text-align:right;"|עשה לך מורה שיוסיף לך זה על זה כזה הדמיון והם א' ג' ט' ומחובר שלשתם י"ג יהיה לראשון א' ולשני ג' ולשלישי ט‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|ועתה שאמר ומחובר שלשתם ס' עשה על זה הדרך | + | :Now that it is said "the sum of what all the three have is 60", do this way: |
| + | |style="text-align:right;"|ועתה שאמר ומחובר שלשתם ס'‫<ref>ס': MS Ithaca om.</ref> עשה על זה הדרך | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> To know how much the first has, say: if, when the sum is 13, the share of the first is 1, now that the sum is 60, how much does he have? | |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{13:1=60:a_1}}</math> |
| + | |style="text-align:right;"|ולדעת כמה יש לראשון אמור אם בהיות המחובר י"ג יהיה החלק הראשון א' עתה שהמחובר ס' כמה יש לו | ||
|- | |- | ||
| | | | ||
| − | : | + | :Say: 60 times 1 is 60. Divide it by 13; it is 4 and 8 parts of 13. |
| + | :<math>\scriptstyle{\color{blue}{a_1=\frac{60\sdot1}{13}=\frac{60}{13}=4+\frac{8}{13}}}</math> | ||
|style="text-align:right;"|אמור ס' פעמי' א' הם ס‫'<br> | |style="text-align:right;"|אמור ס' פעמי' א' הם ס‫'<br> | ||
| − | חלקם בי"ג יהיו ד' וח' חלקים מי"ג | + | חלקם בי"ג יהיו ד' וח'‫<ref>ד' וח': MS Ithaca י"ג וי"א</ref> חלקים מי"ג |
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> To know the share of the second, say: if, when the sum is 13, the share of the second is 3, now that the sum is 60, how much is his share? | |
| − | |style="text-align:right;"|ולדעת חלק | + | :<math>\scriptstyle{\color{blue}{13:3=60:a_2}}</math> |
| + | |style="text-align:right;"|ולדעת חלק השיני אמור אם בהיות המחובר י"ג יהיה החלק‫<ref>החלק: MS Ithaca המחובר</ref> השני ג'‫<ref>ג': MS Ithaca om.</ref> עתה שהמחובר ס' כמה הוא חלקו | ||
|- | |- | ||
| | | | ||
| − | : | + | :Say: 60 times 3 is 180. Divide it by 13; it is 13 and 11 parts of 13. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{a_2=\frac{60\sdot3}{13}=\frac{180}{13}=13+\frac{11}{13}}}</math> |
| − | חלקם בי"ג יהיו י"ג וי"א חלקים מי"ג | + | |style="text-align:right;"|אמו' ס' פעמי' ג' הם ק"פ<br> |
| + | חלקם בי"ג יהיו י"ג וי"א חלקים מי"ג‫<ref>ולדעת חלק השיני... וי"א חלקים מי"ג: MS Ithaca twice</ref> | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> To know the share of the third, say: if, when the sum is 13, the share of the third is 9, now that the sum is 60, how much is his share? | |
| + | :<math>\scriptstyle{\color{blue}{13:9=60:a_3}}</math> | ||
|style="text-align:right;"|ולדעת חלק השלישי אמור אם בהיות המחובר י"ג היה חלק השלישי ט' עתה בהיות המחובר ס' כמה הוא חלקו | |style="text-align:right;"|ולדעת חלק השלישי אמור אם בהיות המחובר י"ג היה חלק השלישי ט' עתה בהיות המחובר ס' כמה הוא חלקו | ||
|- | |- | ||
| | | | ||
| − | : | + | :Say: 60 times 9 is 540. Divide it by 13; it is 41 and 7 parts of 13. |
| + | :<math>\scriptstyle{\color{blue}{a_3=\frac{60\sdot9}{13}=\frac{540}{13}=41+\frac{7}{13}}}</math> | ||
|style="text-align:right;"|אמור ס' פעמי' ט' הם תק"מ<br> | |style="text-align:right;"|אמור ס' פעמי' ט' הם תק"מ<br> | ||
| − | חלקם בי"ג יהיו מ"א וז' חלקים מי"ג | + | חלקם בי"ג יהיו <s>י"א</s> מ"א וז' חלקים מי"ג |
|- | |- | ||
| | | | ||
| − | :Check: <math>\scriptstyle{\color{blue}{\left(4+\frac{8}{13}\right)+\left(13+\frac{11}{13}\right)+\left(41+\frac{7}{13}\right)=60}}</math> | + | :<span style=color:green>'''Check:'''</span> If you sum them up the total is 60. |
| + | :<math>\scriptstyle{\color{blue}{\left(4+\frac{8}{13}\right)+\left(13+\frac{11}{13}\right)+\left(41+\frac{7}{13}\right)=60}}</math> | ||
|style="text-align:right;"|ואם תקבצם יהיו כלם ס‫' | |style="text-align:right;"|ואם תקבצם יהיו כלם ס‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | :The same if they exceed each other by 2, or 4, or 5 and if they are more than three friends, find the denominator in the appropriate way and you will find your wish. |
:<math>\scriptstyle X+aX+a^2X=60</math> | :<math>\scriptstyle X+aX+a^2X=60</math> | ||
|style="text-align:right;"|וכן אם יהיו זה על זה ב' או ד' או ה' וכן אם יהיו יותר מג' חברים כמו כן עשה המורה על דרך הראוי ותמצא חפצך | |style="text-align:right;"|וכן אם יהיו זה על זה ב' או ד' או ה' וכן אם יהיו יותר מג' חברים כמו כן עשה המורה על דרך הראוי ותמצא חפצך | ||
| Line 3,220: | Line 4,248: | ||
:Issachar's share turned out to be equal to a fifth of Reuven's share. | :Issachar's share turned out to be equal to a fifth of Reuven's share. | ||
:Yehuda's share turned out to be equal to a fifth of Shimon's share | :Yehuda's share turned out to be equal to a fifth of Shimon's share | ||
| − | |style="text-align:right;"|יעקב חלק לחמשה בניו ל"ה דינרים<br> | + | |style="text-align:right;"|<big>יעקב חלק לחמשה בניו ל"ה דינרים</big><br> |
והיה חלק ראובן גדול משל לוי ושל לוי גדול משל יששכר<br> | והיה חלק ראובן גדול משל לוי ושל לוי גדול משל יששכר<br> | ||
ושל שמעון כעודף ראובן על לוי<br> | ושל שמעון כעודף ראובן על לוי<br> | ||
| − | ושל יהודה כעודף לוי על יששכר<br> | + | ושל יהודה כעודף לוי על <s>ישכ</s> יששכר<br> |
| − | ואז מצא | + | ואז מצא ישכר בחלקו חמשית של ראובן<br> |
ויהודה מצא בחלקו חמשית של שמעון{{#annotend:6a8i}} | ויהודה מצא בחלקו חמשית של שמעון{{#annotend:6a8i}} | ||
|- | |- | ||
| − | | | + | | colspan="2"| |
:<math>\scriptstyle\begin{cases}\scriptstyle a_1+a_2+a_3+a_4+a_5=35\\\scriptstyle a_2=a_1-a_3\\\scriptstyle a_5=a_3-a_4\\\scriptstyle a_4=\frac{1}{5}a_1\\\scriptstyle a_5=\frac{1}{5}a_2\end{cases}</math> | :<math>\scriptstyle\begin{cases}\scriptstyle a_1+a_2+a_3+a_4+a_5=35\\\scriptstyle a_2=a_1-a_3\\\scriptstyle a_5=a_3-a_4\\\scriptstyle a_4=\frac{1}{5}a_1\\\scriptstyle a_5=\frac{1}{5}a_2\end{cases}</math> | ||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :According to this, Reuven's share:= is 15. |
| − | |style="text-align:right;"|והנה לפי זה חלק ראובן ט"ו | + | :<math>\scriptstyle{\color{blue}{a_1=15}}</math> |
| + | |style="text-align:right;"|והנה ‫<ref>39r</ref>לפי זה חלק ראובן ט"ו | ||
|- | |- | ||
| | | | ||
| − | : | + | :Levi's share is 5. |
| + | :<math>\scriptstyle{\color{blue}{a_3=5}}</math> | ||
|style="text-align:right;"|וחלק לוי ה‫' | |style="text-align:right;"|וחלק לוי ה‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | :Issachar's share is 3. |
| + | :<math>\scriptstyle{\color{blue}{a_4=3}}</math> | ||
|style="text-align:right;"|וחלק יששכר ג‫' | |style="text-align:right;"|וחלק יששכר ג‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | :Shimon's share is 10. |
| + | :<math>\scriptstyle{\color{blue}{a_2=10}}</math> | ||
|style="text-align:right;"|וחלק שמעון י‫' | |style="text-align:right;"|וחלק שמעון י‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | :Yehuda's share is 2. |
| + | :<math>\scriptstyle{\color{blue}{a_5=2}}</math> | ||
|style="text-align:right;"|וחלק יהודה ב‫' | |style="text-align:right;"|וחלק יהודה ב‫' | ||
|- | |- | ||
| | | | ||
| − | ::Check: <math>\scriptstyle{\color{blue}{a_1+a_2+a_3+a_4+a_5=35}}</math> | + | :<span style=color:green>'''Check:'''</span> The total is 35. |
| + | :<math>\scriptstyle{\color{blue}{a_1+a_2+a_3+a_4+a_5=35}}</math> | ||
|style="text-align:right;"|וכך כלם ל"ה | |style="text-align:right;"|וכך כלם ל"ה | ||
|- | |- | ||
| | | | ||
| − | : | + | *The one who asks says: Jacob gave his five sons 60 dinar according to the previous problem. |
| − | |style="text-align:right;"|והנה אמר השואל יעקב חלק לה' בניו ס' דינרי' כפי השאלה הקודמ' עשה כפי זה הדרך | + | |style="text-align:right;"|והנה אמר השואל יעקב חלק לה' בניו ס' דינרי' כפי השאלה הקודמ‫' |
| + | |- | ||
| + | | | ||
| + | :Do as follows: | ||
| + | |style="text-align:right;"|עשה כפי זה הדרך | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> If you want to find Reuven's share, say: if, when the amount of money is 35 dinar, Reuven's share is 15, when the amount of money is 60, how much is his share? | |
| − | |style="text-align:right;"|אם תרצה לדעת חלק ראובן אמור אם בהיות סכום המעות ל"ה היה חלק ראובן ט"ו בהיות סכום הדינרי' ס' כמה הוא חלקו | + | :<math>\scriptstyle{\color{blue}{35:15=60:a_1}}</math> |
| + | |style="text-align:right;"|אם תרצה לדעת חלק ראובן אמור אם בהיות סכום המעות ל"ה היה חלק ראובן ט"ו בהיות סכום‫<ref>ל"ה... בהיות סכום: MS Ithaca om.</ref> הדינרי' ס' כמה הוא חלקו | ||
|- | |- | ||
| | | | ||
| − | :: | + | :Say: 60 times 15 is 900. Divide it by 35; it is 25 and 25 parts of 35. |
| − | |style="text-align:right;"|אמור ס' פעמים ט"ו הם תת"ק<br> | + | :<math>\scriptstyle{\color{blue}{a_1=\frac{60\sdot15}{35}=\frac{900}{35}=25+\frac{25}{35}}}</math> |
| + | |style="text-align:right;"|אמור ס' פעמים ט"ו‫<ref>ט"ו: MS Ithaca om.</ref> הם תת"ק<br> | ||
חלקם בל"ה יהיו כ"ה וכ"ה חלקים מל"ה | חלקם בל"ה יהיו כ"ה וכ"ה חלקים מל"ה | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> To find Levi's share, say: if, when the amount of money is 35 dinar, Levi's share is 5, when the amount of money is [60], how much is [his share]? | |
| + | :<math>\scriptstyle{\color{blue}{35:5=60:a_3}}</math> | ||
|style="text-align:right;"|ולדעת חלק לוי אמור אם בהיות סכום הדינרי' ל"ה היה חלק לוי ה' בהיות סכום המעות ח' כמה יהיו | |style="text-align:right;"|ולדעת חלק לוי אמור אם בהיות סכום הדינרי' ל"ה היה חלק לוי ה' בהיות סכום המעות ח' כמה יהיו | ||
|- | |- | ||
| | | | ||
| − | :: | + | :Say: 60 times 5 is 300. Divide it by 35; it is 8 and 20 parts of 35. |
| − | |style="text-align:right;"|אמור ס' פעמי' ה' הם ש‫'<br> | + | :<math>\scriptstyle{\color{blue}{a_3=\frac{60\sdot5}{35}=\frac{300}{35}=8+\frac{20}{35}}}</math> |
| + | |style="text-align:right;"|אמור ס' פעמי' ה'‫<ref>ה': MS Ithaca om.</ref> הם ש‫'<br> | ||
חלקם בל"ה יהיו ח' דינרי' וכ' חלקים מל"ה | חלקם בל"ה יהיו ח' דינרי' וכ' חלקים מל"ה | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> To find Issachar's share, say: if, when the amount of money is 35 dinar, his share is 3, when the amount of money is 60, how much is [his share]? | |
| − | |style="text-align:right;"|ולדעת חלק יששכר אמור אם בהיות סך המעות ל"ה דינרי' היה חלקו ג' בהיות סך המעות ס' כמה יהיו | + | :<math>\scriptstyle{\color{blue}{35:3=60:a_4}}</math> |
| + | |style="text-align:right;"|ולדעת חלק יששכר אמור אם בהיות סך המעות ל"ה דינרי' היה חלקו ג'‫<ref>ג': MS Ithaca om.</ref> בהיות סך המעות ס' כמה יהיו | ||
|- | |- | ||
| | | | ||
| − | :: | + | :Say: 60 times 3 is 180. Divide it by 35; it is 5 and 5 parts of 35. |
| + | :<math>\scriptstyle{\color{blue}{a_4=\frac{60\sdot3}{35}=\frac{180}{35}=5+\frac{5}{35}}}</math> | ||
|style="text-align:right;"|אמור ס' פעמים ג' הם ק"פ<br> | |style="text-align:right;"|אמור ס' פעמים ג' הם ק"פ<br> | ||
חלקם בל"ה יהיו ה' וה' חלקים מל"ה | חלקם בל"ה יהיו ה' וה' חלקים מל"ה | ||
|- | |- | ||
| | | | ||
| − | : | + | :To find Shimon's share, which is as the excess of Reuven's [share] over Levi's [share]: his share is 17 and 5 parts of 35. |
| − | |style="text-align:right;"|ולדעת חלק שמעון שהוא | + | :<math>\scriptstyle{\color{blue}{a_2=a_1-a_3={\color{OliveGreen}{\left(25+\frac{25}{35}\right)-\left(8+\frac{20}{35}\right)}}=17+\frac{5}{35}}}</math> |
| + | |style="text-align:right;"|ולדעת חלק [שמעון שהוא כעודף ראובן על לוי יהיה חלקו י"ז וה' חלקים מל"ה | ||
|- | |- | ||
| | | | ||
| − | : | + | :To find Yehuda's share, which is as the excess of Levi's [share] over Issachar's [share]: his share is 3 and 15 parts of 35. |
| − | |style="text-align:right;"|ולדעת חלק יהודה שהוא | + | :<math>\scriptstyle{\color{blue}{a_5=a_3-a_4={\color{OliveGreen}{\left(8+\frac{20}{35}\right)-\left(5+\frac{5}{35}\right)}}=3+\frac{15}{35}}}</math> |
| + | |style="text-align:right;"|ולדעת חלק]‫<ref>MS Ithaca om.</ref> יהודה שהוא כעודף לוי על יששכר יהיו חלקו ג' וט"ו חלקי' מל"ה | ||
|- | |- | ||
| | | | ||
| − | ::Check: | + | :<span style=color:green>'''Check:'''</span> If you sum up all the numbers, it is 60. You find that Issachar's share is a fifth of Reuven's share and Yehuda's share is a fifth of Shimon's share. |
| − | |||
|style="text-align:right;"|ואם תקבץ כל המספרים יהיו ס‫'<br> | |style="text-align:right;"|ואם תקבץ כל המספרים יהיו ס‫'<br> | ||
| − | + | ותמצ' [חלק]‫<ref>MS Ithaca om.</ref> <s>ישכ</s> יששכר חומש חלק ראובן<br> | |
ויהודה מצא חלקו חמישית חלק שמעון | ויהודה מצא חלקו חמישית חלק שמעון | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | :<math>\scriptstyle{\color{blue}{\begin{cases}\scriptstyle a_1+a_2+a_3+a_4+a_5=60\\\scriptstyle a_4=\frac{1}{5}a_1\\\scriptstyle a_5=\frac{1}{5}a_2\end{cases}}}</math> | ||
|- | |- | ||
| | | | ||
| − | === Joint Purchase Problem - If You Give Me - Amounts of Money === | + | === <span style=color:green>Joint Purchase Problem - If You Give Me - Amounts of Money</span> === |
| | | | ||
| Line 3,312: | Line 4,359: | ||
:The second answered: all what is in my purse and a third of what both of you have will be 60. | :The second answered: all what is in my purse and a third of what both of you have will be 60. | ||
:The third replied: all what is in my purse and a quarter of what both of you have will be 60. | :The third replied: all what is in my purse and a quarter of what both of you have will be 60. | ||
| − | :How much money did each of them have? | + | :How much money did each of them have? |
| − | + | |style="text-align:right;"|<big>שלשה חברים אומר הראשון לשני כל מה</big> שבכיסי וחצי שניכם עולה ס‫'<br> | |
| − | |style="text-align:right;"|שלשה חברים אומר הראשון לשני כל מה שבכיסי וחצי שניכם עולה ס‫'<br> | ||
ענה השיני כל מה שבכיסי ושליש שניכם עולה ס‫'<br> | ענה השיני כל מה שבכיסי ושליש שניכם עולה ס‫'<br> | ||
ענה השלישי כל מה שבכיסי ורביע שניכם עולה ס‫'<br> | ענה השלישי כל מה שבכיסי ורביע שניכם עולה ס‫'<br> | ||
כמה מעות היו לכל אחד ואחד{{#annotend:htUo}} | כמה מעות היו לכל אחד ואחד{{#annotend:htUo}} | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle X+\frac{1}{2}Y+\frac{1}{2}Z=60\\\scriptstyle Y+\frac{1}{3}X+\frac{1}{3}Z=60\\\scriptstyle Z+\frac{1}{4}X+\frac{1}{4}Y=60\end{cases}</math> | ||
| + | |- | ||
| + | | | ||
| + | :<span style=color:green>'''False Position:'''</span> We find three numbers, which are 5, 11, 13 that match the question. | ||
| + | :<math>\scriptstyle{\color{blue}{X_1=5\quad Y_1=11\quad Z_1=13}}</math> | ||
| + | |style="text-align:right;"|מצאנו ג' מספרי' והם ה' י"א י"ג שהם כעין השאילה הזאת | ||
|- | |- | ||
| | | | ||
| − | :: | + | :Because, 5 says to 11 and 13: me and a half of both of you are 17. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{5+\left(\frac{1}{2}\sdot11\right)+\left(\frac{1}{2}\sdot13\right)=17}}</math> |
| + | |style="text-align:right;"|כי ה' אומ' לי"א ולי"ג אני וחצי שניכם י"ז | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{ | + | :11 says to 13 and 5: me and a third of both of you are 17. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{11+\left(\frac{1}{3}\sdot5\right)+\left(\frac{1}{3}\sdot13\right)=17}}</math> |
| − | וי"א אומ' לי"ג וה' | + | |style="text-align:right;"|וי"א אומ' לי"ג [וה']‫<ref>MS Ithaca om.</ref> אני ושליש שניכם י"ז |
| − | |||
|- | |- | ||
| | | | ||
| + | :13 says to 11 and 5: me and a quarter of both of you are 17. | ||
| + | :<math>\scriptstyle{\color{blue}{13+\left(\frac{1}{4}\sdot5\right)+\left(\frac{1}{4}\sdot11\right)=17}}</math> | ||
| + | |style="text-align:right;"|י"ג אומרת לי"א ולה' אני ורביע שניכם י"ז | ||
| + | |- | ||
| + | | | ||
| + | :You see that when it is said "the sum of the three of us is 17", the first has 5, the second has 11 and the third has 13. Now that it is said "the sum of the three of us is 60", we relate: | ||
|style="text-align:right;"|והנך רואה שאמרו מחובר שלשתינו י"ז יהיה לאחד ה' ולשני י"א ולשלישי י"ג ועכשיו שאמרו מחובר שלשתינו ס' נשיב לערכים | |style="text-align:right;"|והנך רואה שאמרו מחובר שלשתינו י"ז יהיה לאחד ה' ולשני י"א ולשלישי י"ג ועכשיו שאמרו מחובר שלשתינו ס' נשיב לערכים | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> We say: as the ratio of 5 to 17, so is the ratio of the first, who asks his friends for a half, to 60. | |
| + | :<math>\scriptstyle{\color{blue}{5:17=X:60}}</math> | ||
|style="text-align:right;"|ונאמר כערך ה' לי"ז כן ערך ראשון לס' הוא ששאל לחביריו החצי | |style="text-align:right;"|ונאמר כערך ה' לי"ז כן ערך ראשון לס' הוא ששאל לחביריו החצי | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> As the ratio of 11 to 17, so is the ratio of the middle, who asks his friends for a third, to 60. | |
| − | |style="text-align:right;"|וכערך י"א לי"ז כן ערך האמצעי לס' הוא ששאל לחבירו השליש | + | :<math>\scriptstyle{\color{blue}{11:17=Y:60}}</math> |
| + | |style="text-align:right;"|וכערך י"א לי"ז כן ערך האמצעי [לס']‫<ref>MS Ithaca ראשון ס'</ref> הוא ששאל לחבירו השליש | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> As the ratio of 13 to 17, so is the ratio of the greater, who asks his friends for a quarter, to 60. | |
| − | |style="text-align:right;"|וכערך י"ג לי"ז כן ערך הגדול אל ס' והוא ששאל | + | :<math>\scriptstyle{\color{blue}{13:17=Z:60}}</math> |
| + | |style="text-align:right;"|וכערך י"ג לי"ז כן ערך הגדול אל ס' והוא ששאל לחביריו הרביע | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|וכן | + | :Relate all the amounts the three mentioned like this: |
| + | |style="text-align:right;"|וכן תעריך לכל הסכומים שיאמרו שלשתם | ||
|- | |- | ||
| | | | ||
| − | + | |style="text-align:right;"|תשיב המורה ה' י"א י"ג | |
| − | |||
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{X=\frac{60\sdot5}{17}=\frac{300}{17}=17+\frac{11}{17}}}</math> | + | :The proportion method is as follows: |
| + | |style="text-align:right;"|ודרך הערכים הוא בעניין הזה | ||
| + | |- | ||
| + | | | ||
| + | :<span style=color:green>'''Rule of Three:'''</span> If you want to know the share of the smaller, say: if, when the sum is 17, the share of the smaller is 5, how much is his share, when the sum is 60? | ||
| + | :<math>\scriptstyle{\color{blue}{17:5=60:X}}</math> | ||
| + | |style="text-align:right;"|‫[אם תרצה לדעת חלק הקטון אמור אם בעת שהמחובר י"ז יהיה חלק הקטן ה' בהיות המחובר ס' כמה הם חלקו | ||
| + | |- | ||
| + | | | ||
| + | :Say: 60 times 5 is 300. Divide it by 17; it is 17 and 11 parts of 17. | ||
| + | :<math>\scriptstyle{\color{blue}{X=\frac{60\sdot5}{17}=\frac{300}{17}=17+\frac{11}{17}}}</math> | ||
|style="text-align:right;"|אמור ס' פעמי' ה' הם ש‫'<br> | |style="text-align:right;"|אמור ס' פעמי' ה' הם ש‫'<br> | ||
חלקם בי"ז יהיו י"ז וי"א חלקים מי"ז | חלקם בי"ז יהיו י"ז וי"א חלקים מי"ז | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> To know the share of the middle, say: if, when the sum is 17, the share of the middle is 11, how much is his share, when the sum is 60? | |
| − | + | :<math>\scriptstyle{\color{blue}{17:11=60:Y}}</math> | |
|style="text-align:right;"|ולדעת חלק האמצעי אמור אם בעת שהמחבר י"ז שוה החלק האמצעי י"א בהיות המחבר ס' כמה הוא חלקו | |style="text-align:right;"|ולדעת חלק האמצעי אמור אם בעת שהמחבר י"ז שוה החלק האמצעי י"א בהיות המחבר ס' כמה הוא חלקו | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{Y=\frac{60\sdot11}{17}=\frac{660}{17}=38+\frac{14}{17}}}</math> | + | :Say: 60 times 11 is 660. Divide it by 17; it is 38 and 14 parts of 17. |
| + | :<math>\scriptstyle{\color{blue}{Y=\frac{60\sdot11}{17}=\frac{660}{17}=38+\frac{14}{17}}}</math> | ||
|style="text-align:right;"|אמור ס' פעמי' י"א הם תר"ס<br> | |style="text-align:right;"|אמור ס' פעמי' י"א הם תר"ס<br> | ||
חלקם בי"ז יהיו ל"ח וי"ד חלקים מי"ז | חלקם בי"ז יהיו ל"ח וי"ד חלקים מי"ז | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> To know the share of the greater, say: if, when the sum is 17, the share of the greater is 13, how much is his share, when the sum is 60? | |
| − | + | :<math>\scriptstyle{\color{blue}{17:13=60:Z}}</math> | |
|style="text-align:right;"|ולדעת חלק הגדול אמור אם בעת שהמחבר הוא י"ז יהיה חלק הגדול י"ג בהיות המחובר ס' כמה הוא חלקו | |style="text-align:right;"|ולדעת חלק הגדול אמור אם בעת שהמחבר הוא י"ז יהיה חלק הגדול י"ג בהיות המחובר ס' כמה הוא חלקו | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{Z=\frac{60\sdot13}{17}=\frac{780}{17}=45+\frac{15}{17}}}</math> | + | :Say: 60 times 13 is 780. Divide it by 17; it is 45 and 15 parts of 17. |
| + | :<math>\scriptstyle{\color{blue}{Z=\frac{60\sdot13}{17}=\frac{780}{17}=45+\frac{15}{17}}}</math> | ||
|style="text-align:right;"|אמור ס' פעמי' י"ג הם תש"פ<br> | |style="text-align:right;"|אמור ס' פעמי' י"ג הם תש"פ<br> | ||
חלקם בי"ז יהיו מ"ה וט"ו חלקים מי"ז | חלקם בי"ז יהיו מ"ה וט"ו חלקים מי"ז | ||
|- | |- | ||
| | | | ||
| + | :The order of the matter is as follows: | ||
| + | |style="text-align:right;"|ויהיה סדר העניין כן | ||
| + | |- | ||
| + | | | ||
| + | :The smaller says: I am 17 and 11 parts of 17, with half the middle, which is 19 and 7 parts of 17, and with half the greater, which is 22 and 16 parts of 17 - the sum of the three of us is 60. | ||
| + | |style="text-align:right;"|אומר הקטן אני שאני י"ז וי"א חלקים מי"ז וחצי האמצעי שהם י"ט וז' חלקים מי"ז וחצי הגדול שהם כ"ב וי"ו חלקים מי"ז מחובר שלשתינו עולה ס‫' | ||
| + | |- | ||
| + | | colspan="2"| | ||
:<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle X+\frac{1}{2}Y+\frac{1}{2}Z &\scriptstyle=\left(17+\frac{11}{17}\right)+\left[\frac{1}{2}\sdot\left(38+\frac{14}{17}\right)\right]+\left[\frac{1}{2}\sdot\left(45+\frac{15}{17}\right)\right]\\&\scriptstyle=\left(17+\frac{11}{17}\right)+\left(19+\frac{7}{17}\right)+\left(22+\frac{16}{17}\right)=60\\\end{align}}}</math> | :<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle X+\frac{1}{2}Y+\frac{1}{2}Z &\scriptstyle=\left(17+\frac{11}{17}\right)+\left[\frac{1}{2}\sdot\left(38+\frac{14}{17}\right)\right]+\left[\frac{1}{2}\sdot\left(45+\frac{15}{17}\right)\right]\\&\scriptstyle=\left(17+\frac{11}{17}\right)+\left(19+\frac{7}{17}\right)+\left(22+\frac{16}{17}\right)=60\\\end{align}}}</math> | ||
| − | |||
|- | |- | ||
| | | | ||
| + | :The middle says: I am 38 and 14 parts of 17, with a third of the smaller, which is 5 and 15 parts of 17, and with a third of the greater, which is 15 and 5 parts of 17 - the sum of the three of us is 60. | ||
| + | |style="text-align:right;"|ואומר האמצעי אני שאני ל"ח וי"ד חלקים מי"ז ושליש הקטן שהם ה' וט"ו חלקים מי"ז ושליש הגדול שהם ט"ו וה' חלקים מי"ז מחובר שלשתינו עולה שהם ס‫' | ||
| + | |- | ||
| + | | colspan="2"| | ||
:<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle Y+\frac{1}{3}X+\frac{1}{3}Z &\scriptstyle=\left(38+\frac{14}{17}\right)+\left[\frac{1}{3}\sdot\left(17+\frac{11}{17}\right)\right]+\left[\frac{1}{3}\sdot\left(45+\frac{15}{17}\right)\right]\\&\scriptstyle=\left(38+\frac{14}{17}\right)+\left(5+\frac{15}{17}\right)+\left(15+\frac{5}{17}\right)=60\\\end{align}}}</math> | :<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle Y+\frac{1}{3}X+\frac{1}{3}Z &\scriptstyle=\left(38+\frac{14}{17}\right)+\left[\frac{1}{3}\sdot\left(17+\frac{11}{17}\right)\right]+\left[\frac{1}{3}\sdot\left(45+\frac{15}{17}\right)\right]\\&\scriptstyle=\left(38+\frac{14}{17}\right)+\left(5+\frac{15}{17}\right)+\left(15+\frac{5}{17}\right)=60\\\end{align}}}</math> | ||
| − | |||
|- | |- | ||
| | | | ||
| + | :The greater says: I am 45 and 15 parts of 17, with a quarter of the smaller, which is 4 and 7 parts of 17, and with a quarter of the middle, which is 9 and 12 parts of 17 - the sum of the three of us is 60. | ||
| + | |style="text-align:right;"|ואמר הגדול אני שאני מ"ה וט"ו חלקים מי"ז רביע הקטן שהוא ד' וז' חלקים מי"ז ורביע האמצעי שהוא ט' וי"ב חלקים מי"ז מחובר שלשתינו עולה ס‫']‫<ref>MS Ithaca om.</ref> | ||
| + | |- | ||
| + | | colspan="2"| | ||
:<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle Z+\frac{1}{4}X+\frac{1}{4}Y &\scriptstyle=\left(45+\frac{15}{17}\right)+\left[\frac{1}{4}\sdot\left(17+\frac{11}{17}\right)\right]+\left[\frac{1}{4}\sdot\left(38+\frac{14}{17}\right)\right]\\&\scriptstyle=\left(45+\frac{15}{17}\right)+\left(4+\frac{7}{17}\right)+\left(9+\frac{12}{17}\right)=60\\\end{align}}}</math> | :<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle Z+\frac{1}{4}X+\frac{1}{4}Y &\scriptstyle=\left(45+\frac{15}{17}\right)+\left[\frac{1}{4}\sdot\left(17+\frac{11}{17}\right)\right]+\left[\frac{1}{4}\sdot\left(38+\frac{14}{17}\right)\right]\\&\scriptstyle=\left(45+\frac{15}{17}\right)+\left(4+\frac{7}{17}\right)+\left(9+\frac{12}{17}\right)=60\\\end{align}}}</math> | ||
| − | |||
|- | |- | ||
| | | | ||
| − | === Multiple Quantities Problems === | + | === <span style=color:green>Multiple Quantities Problems</span> === |
| + | |||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | ==== <span style=color:green>Three Brothers - Money</span> ==== | ||
| | | | ||
| Line 3,403: | Line 4,495: | ||
:Then their shares were equal. | :Then their shares were equal. | ||
:How much was the share of each of them at first? | :How much was the share of each of them at first? | ||
| − | + | |style="text-align:right;"|<big>שאלה יעקב חלק [כ"ד]‫<ref>MS Ithaca עשרים</ref> דינרים לג' בניו</big><br> | |
| − | |style="text-align:right;"|שאלה יעקב חלק כ"ד דינרים לג' בניו<br> | ||
והיה חלק ראובן גדול משל שמעון וחלק שמעון גדול מחלק לוי<br> | והיה חלק ראובן גדול משל שמעון וחלק שמעון גדול מחלק לוי<br> | ||
נתן ראובן לשני אחיו מחלקו כפי מה שהיה חלקם<br> | נתן ראובן לשני אחיו מחלקו כפי מה שהיה חלקם<br> | ||
| Line 3,411: | Line 4,502: | ||
ואז נמצא חלק שלשתם שוה<br> | ואז נמצא חלק שלשתם שוה<br> | ||
כמה היה חלק כל אחד מהם בראשונה{{#annotend:87qX}} | כמה היה חלק כל אחד מהם בראשונה{{#annotend:87qX}} | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle a_1+a_2+a_3=24\\\scriptstyle2\sdot\left[2\sdot\left[a_1-\left(a_2+a_3\right)\right]\right]\\\scriptstyle=2\sdot\left[\left(2\sdot a_2\right)-\left[\left[a_1-\left(a_2+a_3\right)\right]+\left(2\sdot a_3\right)\right]\right]\\\scriptstyle=\left[2\sdot\left(2\sdot a_3\right)\right]-\left[\left[2\sdot\left[a_1-\left(a_2+a_3\right)\right]\right]+\left[\left(2\sdot a_2\right)-\left[\left[a_1-\left(a_2+a_3\right)\right]+\left(2\sdot a_3\right)\right]\right]\right]\end{cases}</math> | ||
| + | |- | ||
| + | | | ||
| + | :Say that the part of the first is 4; the part of the second is 7; the part of the third is 13. | ||
| + | |style="text-align:right;"|אמור כי החלק האחד היה ד' והחלק השני ז' והחלק השלישי י"ג | ||
|- | |- | ||
| | | | ||
| − | : | + | :We find that at the end the share of each is 8 equally. |
| − | + | |style="text-align:right;"|ונמצא באחרונה חלק כל אחד מהם ח' שוה בשוה | |
| − | |style="text-align:right;"| | ||
| − | |||
| − | |||
| − | ונמצא באחרונה חלק כל אחד מהם ח' שוה בשוה | ||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :The Reuven's share was 13 [<math>\scriptstyle{\color{blue}{a_1=13}}</math>]; Shimon's share was 7 [<math>\scriptstyle{\color{blue}{a_2=7}}</math>]; and Levi's share was 4 [<math>\scriptstyle{\color{blue}{a_3=4}}</math>]. |
| − | + | |style="text-align:right;"|והיה חלק ראובן י"ג וחלק שמעו' ז' וחלק לוי ד‫' | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | : | + | :Reuven started and gave 7 of his 13 to Shimon as his share and 4 to Levi as his share. |
| − | + | :<math>\scriptstyle{\color{blue}{a_1-\left(a_2+a_3\right)=13-\left(7+4\right)}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והנה התחיל ראובן ונתן מן הי"ג שלו ז' לשמעון כפי חלקו וד' [ל]לוי כפי חלקו |
|- | |- | ||
| | | | ||
| − | : | + | :Shimon, whose share is 14 now, gave 2 to Reuven as his share and 8 to Levi as his share. |
| − | + | :<math>\scriptstyle{\color{blue}{\left(2\sdot a_2\right)-\left[\left[a_1-\left(a_2+a_3\right)\right]+\left(2\sdot a_3\right)\right]=14-\left(2+8\right)}}</math> | |
| + | |style="text-align:right;"|‫[ושמעון שחלקו מעתה הם י"ד נתן ב' לראובן כפי חלקו וח' ללוי כפי חלקו]‫<ref>MS Ithaca om.</ref> | ||
| + | |- | ||
| + | | | ||
| + | :Levi, whose share is 16 now, gave 4 to Reuven and 4 to Shimon. | ||
|style="text-align:right;"|ולוי שחלקו הם מעתה י"ו נתן ד' לראובן וד' לשמעון | |style="text-align:right;"|ולוי שחלקו הם מעתה י"ו נתן ד' לראובן וד' לשמעון | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | :<math>\scriptstyle{\color{blue}{\begin{align}&\scriptstyle\left[2\sdot\left(2\sdot a_3\right)\right]-\left[\left[2\sdot\left[a_1-\left(a_2+a_3\right)\right]\right]+\left[\left(2\sdot a_2\right)-\left[\left[a_1-\left(a_2+a_3\right)\right]+\left(2\sdot a_3\right)\right]\right]\right]\\&\scriptstyle=16-\left(4+4\right)\\\end{align}}}</math> | ||
|- | |- | ||
| | | | ||
| − | : | + | :Now, each has 8. |
|style="text-align:right;"|מעתה יש לכל אחד ואחד מהם ח‫' | |style="text-align:right;"|מעתה יש לכל אחד ואחד מהם ח‫' | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== <span style=color:green>Selling Cloth</span> ==== | ||
| + | |||
| + | | | ||
|- | |- | ||
| | | | ||
| Line 3,444: | Line 4,549: | ||
:One sold one cubit for 4 dinar, the second sold one cubit for 5 dinar, and the third sold one cubit for 6 dinar. | :One sold one cubit for 4 dinar, the second sold one cubit for 5 dinar, and the third sold one cubit for 6 dinar. | ||
:All of them earned the same amount of money. | :All of them earned the same amount of money. | ||
| − | :How many cubits of cloth did each of them sell and how much money did each of them get? | + | :How many cubits of cloth did each of them sell and how much money did each of them get? |
| − | + | |style="text-align:right;"|<big>אדם אחד נתן לשלש[ת] [בניו]</big>‫<ref>MS Ithaca illegible</ref> ‫<ref>39v</ref>שלשי' אמות בגד למכור הלכו בשוק למכרו<br> | |
| − | |style="text-align:right;"|אדם אחד נתן | ||
האחד מכר בד' דינרי' האמה והשני מכר בה' דינרי' האמה והשלישי מכר בו' דינרי' האמה<br> | האחד מכר בד' דינרי' האמה והשני מכר בה' דינרי' האמה והשלישי מכר בו' דינרי' האמה<br> | ||
וכלם הביאו מעות בשוה<br> | וכלם הביאו מעות בשוה<br> | ||
כמה מעות בגד מכר אחד כל אחד וכמה מעות הביא כל אחד{{#annotend:PpNo}} | כמה מעות בגד מכר אחד כל אחד וכמה מעות הביא כל אחד{{#annotend:PpNo}} | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle a_1+a_2+a_3=30\\\scriptstyle4a_1=5a_2=6a_3\end{cases}</math> | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''False Position:'''</span> First say: a quarter, a fifth, and a sixth are found in 60. | |
|style="text-align:right;"|אמור תחלה רביע וחומש ושתות ימצאו בס‫' | |style="text-align:right;"|אמור תחלה רביע וחומש ושתות ימצאו בס‫' | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Denominator:'''</span> The quarter is 15; the fifth is 12; the sixth is 10; their sum is 37 and this is the denominator. | |
| + | :<math>\scriptstyle{\color{blue}{\left(\frac{1}{4}\sdot60\right)+\left(\frac{1}{5}\sdot60\right)+\left(\frac{1}{6}\sdot60\right)=15+12+10=37}}</math> | ||
|style="text-align:right;"|הרביע הוא ט"ו והחומש הוא י"ב והשתות י' וכללם ל"ז והוא המחלק | |style="text-align:right;"|הרביע הוא ט"ו והחומש הוא י"ב והשתות י' וכללם ל"ז והוא המחלק | ||
|- | |- | ||
| | | | ||
| − | : | + | :If you want to know how much cloth sold the one who sold one cubit for 4 dinar: |
| − | ::<span style=color: | + | |style="text-align:right;"|ואם תרצה לדעת כמה בגד מכר אותו שמכר בד' דינרי' האמה |
| − | |style="text-align:right;"| | + | |- |
| + | | | ||
| + | :<span style=color:green>'''Rule of Three:'''</span> Say: the quarter of 60 is 15. Multiply it by the measure of the cloth, which is 30 cubits, and say: 15 times 30 is 450. Divide it by 37, which is the denominator; the result is 12 cubits and 6 parts of 37 of one cubit. | ||
| + | :<math>\scriptstyle{\color{blue}{a_1=\frac{\left(\frac{1}{4}\sdot60\right)\sdot30}{37}=\frac{15\sdot30}{37}=\frac{450}{37}=12+\frac{6}{37}}}</math> | ||
| + | |style="text-align:right;"|אמור הרביע מט"ו מס' הוא ט"ו ותרבה אותו על סך הבגד שהו' ל' אמות ואמור ט"ו פעמים ל' הם ת"נ<br> | ||
חלקם בל"ז שהוא המחלק יבאו י"ב אמות וו' חלקי' מל"ז מן האמה | חלקם בל"ז שהוא המחלק יבאו י"ב אמות וו' חלקי' מל"ז מן האמה | ||
|- | |- | ||
| | | | ||
| − | : | + | :If you want to know how much money received the one who sold one cubit for 4 dinar: |
|style="text-align:right;"|ואם תרצה לדעת כמה מעות קבל זה שמכר בד' דינרי' האמה | |style="text-align:right;"|ואם תרצה לדעת כמה מעות קבל זה שמכר בד' דינרי' האמה | ||
|- | |- | ||
| | | | ||
| − | :::<math>\scriptstyle{\color{blue}{4\sdot12=48}}</math> | + | :Say: for 12 cubits he received 48 dinar. |
| + | :<math>\scriptstyle{\color{blue}{4\sdot12=48}}</math> | ||
|style="text-align:right;"|אמור מי"ב אמות קבל מ"ח דינרי‫' | |style="text-align:right;"|אמור מי"ב אמות קבל מ"ח דינרי‫' | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> For the 6 parts of 37 do as follows: if 37, which is a whole cubit, is worth 48 pešiṭim, which is 4 dinar, how much are 6 parts of 37 worth? | |
| + | :<math>\scriptstyle{\color{blue}{37:48=6:X}}</math> | ||
|style="text-align:right;"|ומן הו' חלקים מל"ז עשה על זה הדרך אמור אם ל"ז שהם אמה שלמה שוה מ"ח פשיטי' שהם ד' דינרי' הו' חלקי' מל"ז כמה שוי‫' | |style="text-align:right;"|ומן הו' חלקים מל"ז עשה על זה הדרך אמור אם ל"ז שהם אמה שלמה שוה מ"ח פשיטי' שהם ד' דינרי' הו' חלקי' מל"ז כמה שוי‫' | ||
|- | |- | ||
| | | | ||
| − | :::<math>\scriptstyle{\color{blue}{X=\frac{6\sdot48}{37}=\frac{288}{37}=7+\frac{29}{37}}}</math> | + | :Say: 6 times 48 is 288. Divide it by 37; the result is 7 pešuṭim and 29 parts of 37. |
| + | :<math>\scriptstyle{\color{blue}{X=\frac{6\sdot48}{37}=\frac{288}{37}=7+\frac{29}{37}}}</math> | ||
|style="text-align:right;"|אמור ו' פעמי' מ"ח הם רפ"ח<br> | |style="text-align:right;"|אמור ו' פעמי' מ"ח הם רפ"ח<br> | ||
חלקם בל"ז יבואו ז"פ וכ"ט חלקים מל"ז | חלקם בל"ז יבואו ז"פ וכ"ט חלקים מל"ז | ||
|- | |- | ||
| | | | ||
| − | : | + | :So, he sold 12 cubits and 6 parts of 37. |
| − | :: and | + | :<math>\scriptstyle{\color{blue}{a_1=12+\frac{6}{37}}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|הרי שמכר י"ב אמות וו' חלקי' מל"ז |
| + | |- | ||
| + | | | ||
| + | :He received 48 dinar, 7 pešuṭim and 29 parts of 37. | ||
| + | :<math>\scriptstyle{\color{blue}{4a_1=48+\frac{7+\frac{29}{37}}{12}}}</math> | ||
| + | |style="text-align:right;"|וקיבל מ"ח דינרי' וז"פ וכ"ט חלקי' מל"ז | ||
| + | |- | ||
| + | | | ||
| + | :If you want to know how much cloth sold the one who sold one cubit for 5 dinar: | ||
| + | |style="text-align:right;"|ואם תרצה לדעת כמה בגד מכר אותו שמכר בה' דינרי' האמה | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> Say: the fifth of 60 is 12. Multiply it by the measure of the cloth, which is 30 cubits, and say: 12 times 30 is 360. Divide it by 37, which is the denominator; the result is 9 cubits and 27 parts of 37 of one cubit. | |
| − | + | :<math>\scriptstyle{\color{blue}{a_2=\frac{\left(\frac{1}{5}\sdot60\right)\sdot30}{37}=\frac{12\sdot30}{37}=\frac{360}{37}=9+\frac{27}{37}}}</math> | |
| − | + | |style="text-align:right;"|אמור החומש מס' הוא י"ב תרבה אותם עם סכום הבגד שהם ל' אמות ואמור י"ב פעמים ל' והם ש"ס<br> | |
| − | |style="text-align:right;"| | ||
חלקם בל"ז שהוא המחלק יבואו ט' אמות וכ"ז חלקים מל"ז מן האמה | חלקם בל"ז שהוא המחלק יבואו ט' אמות וכ"ז חלקים מל"ז מן האמה | ||
|- | |- | ||
| | | | ||
| − | : | + | :If you want to know how much money received the one who sold one cubit for 5 dinar: |
|style="text-align:right;"|ואם תרצה לדעת כמה מעות קבל אותו שמכר בה' דינרי' האמה | |style="text-align:right;"|ואם תרצה לדעת כמה מעות קבל אותו שמכר בה' דינרי' האמה | ||
|- | |- | ||
| | | | ||
| − | :::<math>\scriptstyle{\color{blue}{5\sdot9=45}}</math> | + | :Say: for 9 cubits at 5 dinar for one cubit he received 45 dinar. |
| − | |style="text-align:right;"|אמור מט' אמות בה' די' האמה קבל מ"ה דינרי‫' | + | :<math>\scriptstyle{\color{blue}{5\sdot9=45}}</math> |
| + | |style="text-align:right;"|אמור מט' אמות [בה' די' האמה]‫<ref>MS Ithaca om.</ref> קבל מ"ה דינרי‫' | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> For the 27 parts of 37 do as the first way, say: if 37, which is a whole [cubit], is worth 60 pešiṭim, which is 5 dinar, how much are 27 parts of 37 worth? | |
| − | |style="text-align:right;"|ומן הכ"ז חלקים מל"ז עשה על דרך הראשון ואמור אם מל"ז שהוא א' שלם שוי' ס' פשיטי' שהם ה' דינרי' הכ"ז חלקי' מל"ז כמה הם שוי‫' | + | :<math>\scriptstyle{\color{blue}{37:60=27:X}}</math> |
| + | |style="text-align:right;"|ומן ה"ז הכ"ז חלקים מל"ז עשה על דרך הראשון ואמור אם מל"ז שהוא א' שלם שוי' ס' פשיטי' שהם ה' דינרי' הכ"ז חלקי' מל"ז כמה הם שוי‫' | ||
|- | |- | ||
| | | | ||
| − | :::<math>\scriptstyle{\color{blue}{X=\frac{27\sdot60}{37}=\frac{1620}{37}=43+\frac{29}{37}}}</math> | + | :Say: 27 times 60 is 1620. Divide it by 37; the result is 43 pešuṭim and 29 parts of 37. |
| + | :<math>\scriptstyle{\color{blue}{X=\frac{27\sdot60}{37}=\frac{1620}{37}=43+\frac{29}{37}}}</math> | ||
|style="text-align:right;"|אמור כ"ז פעמי' ס' הם אלף ותר"כ<br> | |style="text-align:right;"|אמור כ"ז פעמי' ס' הם אלף ותר"כ<br> | ||
חלקם בל"ז יבואו מ"ג פשיטים וכ"ט חלקי' מל"ז | חלקם בל"ז יבואו מ"ג פשיטים וכ"ט חלקי' מל"ז | ||
|- | |- | ||
| | | | ||
| − | : | + | :Add them to the 45 dinar he received for the 9 cubits of cloth he sold; this is what he sold at 5 dinar for one cubit. |
| − | + | |style="text-align:right;"|‫[וצרף אותם עם המ"ה די' שקבל מט' אמות בגד שמכר הרי שזה שמכר בה' די' האמה | |
| − | |style="text-align:right;"|וצרף אותם עם המ"ה די' שקבל מט' אמות בגד שמכר הרי שזה שמכר בה' די' האמה | ||
|- | |- | ||
| | | | ||
| − | : | + | :So, he sold 9 cubits and 27 parts of 37. |
| − | ::<span style=color: | + | :<math>\scriptstyle{\color{blue}{a_2=9+\frac{27}{37}}}</math> |
| − | + | |style="text-align:right;"|ומכר ט' אמו' וכ"ז חלקים מל"ז | |
| − | |style="text-align:right;"| | + | |- |
| + | | | ||
| + | :He received 48 dinar, 7 pešuṭim and 29 parts of 37, as the first. | ||
| + | :<math>\scriptstyle{\color{blue}{5a_2=48+\frac{7+\frac{29}{37}}{12}}}</math> | ||
| + | |style="text-align:right;"|קבל מ"ח די' וז' פשו' וכ"ט חלקים מל"ז]‫<ref>MS Ithaca om.</ref> כמו הראשון | ||
| + | |- | ||
| + | | | ||
| + | :If you want to know how much cloth sold the one who sold one cubit for 6 dinar: | ||
| + | |style="text-align:right;"|ואם תרצה לדעת כמה בגד מכר אותו שמכר בו' דינרי' האמה | ||
| + | |- | ||
| + | | | ||
| + | :<span style=color:green>'''Rule of Three:'''</span> Say: the sixth of 60 is 10. Multiply it by the measure of the cloth, which is 30 cubits, and say: 10 times 30 is 300. Divide it by 37; it is 8 cubits and 4 parts of 37 of one cubit. | ||
| + | :<math>\scriptstyle{\color{blue}{a_3=\frac{\left(\frac{1}{6}\sdot60\right)\sdot30}{37}=\frac{10\sdot30}{37}=\frac{300}{37}=8+\frac{4}{37}}}</math> | ||
| + | |style="text-align:right;"|אמור [השתות מס' הוא י' תרבה אותם עם סכום הבגד שהם ל' אמות ואמו' י' פעמ' ל' הם ש‫'<br> | ||
חלקם בל"ז יהיו ח' אמות וד' חלקים מל"ז | חלקם בל"ז יהיו ח' אמות וד' חלקים מל"ז | ||
|- | |- | ||
| | | | ||
| − | : | + | :If you want to know how much money received the one who sold one cubit for 6 dinar: |
|style="text-align:right;"|ואם תרצה לדעת כמה מעות קבל זה שמכר בו' די' האמה | |style="text-align:right;"|ואם תרצה לדעת כמה מעות קבל זה שמכר בו' די' האמה | ||
|- | |- | ||
| | | | ||
| − | :::<math>\scriptstyle{\color{blue}{6\sdot8=48}}</math> | + | :Say: for 8 cubits he received 48 dinar. |
| − | |style="text-align:right;"|אמו' מן הח' אמות קבל מ"ח דינרי‫' | + | :<math>\scriptstyle{\color{blue}{6\sdot8=48}}</math> |
| + | |style="text-align:right;"|אמו']‫<ref>MS Ithaca om.</ref> מן הח' אמות קבל מ"ח דינרי‫' | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> For the 4 parts of 37, say: if 37, which is a whole [cubit], is worth 72 pešiṭim, which is 6 dinar, how much are 4 parts of 37 worth? | |
| + | :<math>\scriptstyle{\color{blue}{37:72=4:X}}</math> | ||
|style="text-align:right;"|ומן הד' חלקים מל"ז אמור אם ל"ז שהוא אחד שלם שוה ע"ב פשיטי' שהם ו' דינרי' הד' חלקי' מל"ז כמה הם שוי‫' | |style="text-align:right;"|ומן הד' חלקים מל"ז אמור אם ל"ז שהוא אחד שלם שוה ע"ב פשיטי' שהם ו' דינרי' הד' חלקי' מל"ז כמה הם שוי‫' | ||
|- | |- | ||
| | | | ||
| − | :::<math>\scriptstyle{\color{blue}{X=\frac{4\sdot72}{37}=\frac{288}{37}=7+\frac{29}{37}}}</math> pešuṭim | + | :Say: 4 times 72 is 288. Divide it by 37; the result is 7 pešuṭim and 29 parts of 37. |
| + | :<math>\scriptstyle{\color{blue}{X=\frac{4\sdot72}{37}=\frac{288}{37}=7+\frac{29}{37}}}</math> pešuṭim | ||
|style="text-align:right;"|אמור ד' פעמים ע"ב הוא רפ"ח<br> | |style="text-align:right;"|אמור ד' פעמים ע"ב הוא רפ"ח<br> | ||
| − | חלקם בל"ז יהיו ז | + | חלקם בל"ז יהיו ז"פ וכ"ט חלקים מל"ז |
|- | |- | ||
| | | | ||
| − | :: | + | :So, the one who sold one cubit for 6 dinar sold 8 cubits and 4 parts of 37 [of one cubit]. |
| − | :: and | + | :<math>\scriptstyle{\color{blue}{a_3=8+\frac{4}{37}}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|הרי שזה שמכר בו' דינרי' האמה מכר ח' אמות וד' חלקי' מל"ז אמות |
| + | |- | ||
| + | | | ||
| + | :He received 48 dinar, 7 pešuṭim and 29 parts of 37, as each of his friends. | ||
| + | :<math>\scriptstyle{\color{blue}{6a_3=48+\frac{7+\frac{29}{37}}{12}}}</math> | ||
| + | |style="text-align:right;"|וקבל מ"ח וז"פ וכ"ט חלקי' מל"ז ככל אחד מחבירו | ||
|- | |- | ||
| | | | ||
| − | === Mixture and Alligation Problem === | + | === <span style=color:green>Mixture and Alligation Problem</span> === |
| | | | ||
| Line 3,559: | Line 4,705: | ||
:You want to know: how many carats per ounce all of them will be together? | :You want to know: how many carats per ounce all of them will be together? | ||
:<math>\scriptstyle\frac{\left(2\sdot14\right)+\left(3\sdot16\right)+\left(5\sdot18\right)}{2+3+5}</math> | :<math>\scriptstyle\frac{\left(2\sdot14\right)+\left(3\sdot16\right)+\left(5\sdot18\right)}{2+3+5}</math> | ||
| − | |style="text-align:right;"|הרי שנתת לצורף י' אונקיות זהב לעשות ממנו מעות<br> | + | |style="text-align:right;"|<big>הרי שנתת לצורף י' אונקיות זהב לעשות</big> ממנו מעות<br> |
הב' אונקיות מהם היה זהב מי"ד קראטי האונקי‫'<br> | הב' אונקיות מהם היה זהב מי"ד קראטי האונקי‫'<br> | ||
והג' אונקיו' מהם היה זהב מי"ו קראטי האונקי‫'<br> | והג' אונקיו' מהם היה זהב מי"ו קראטי האונקי‫'<br> | ||
| − | והה' אונקיו' מהם היה זהב מי"ח קראטי האונקי‫'<br> | + | והה' אונקיו' מהם היה זהב מי"ח קראטי [האונקי‫'<br> |
| − | + | והצ]ורף צירף כל הזהב יחד<br> | |
| − | ותרצה לדעת מכמה קראטי יהיה האונק' של | + | ותרצה לדעת מכמה קראטי יהיה [האונק' של הזה]ב שנתערב כלו יחד{{#annotend:pSli}} |
|- | |- | ||
| | | | ||
| − | : | + | :Take the total sum of the ounces of gold; take also the sum of the carat; divide the [sum of] the carat by the sum of the ounces. |
| − | |style="text-align:right;"|ואתה קח סכום כל הא' על האוקיו' של זהב וקח סכום הקראטי ג"כ וחלק הקראטי לסכום האוקיו‫' | + | |style="text-align:right;"|ואתה קח סכום כל הא' ‫<ref>40r</ref>על האוקיו' של זהב וקח סכום הקראטי ג"כ וחלק הקראטי לסכום האוקיו‫' |
|- | |- | ||
| | | | ||
| − | + | :2 ounces of 14 carat are 28 carat. | |
| + | :<math>\scriptstyle{\color{blue}{2\sdot14=28}}</math> | ||
|style="text-align:right;"|כיצד הב' אוקיו' לי"ד קראטי האוקי' עולות כ"ח קראטי | |style="text-align:right;"|כיצד הב' אוקיו' לי"ד קראטי האוקי' עולות כ"ח קראטי | ||
|- | |- | ||
| | | | ||
| − | + | :3 ounces of 16 carat are 48 carat. | |
| + | :<math>\scriptstyle{\color{blue}{3\sdot16=48}}</math> | ||
|style="text-align:right;"|והג' אונקיו' מי"ו קראטי האוקיו' עולי' מ"ח קראטי | |style="text-align:right;"|והג' אונקיו' מי"ו קראטי האוקיו' עולי' מ"ח קראטי | ||
|- | |- | ||
| | | | ||
| − | + | :5 ounces of 18 carat are 90 carat. | |
| − | |style="text-align:right;"|והה' אונקיו' מי"ח קראטי עולות צ' | + | :<math>\scriptstyle{\color{blue}{5\sdot18=90}}</math> |
| + | |style="text-align:right;"|והה' אונקיו' מי"ח קראטי עולות צ' קאראטי | ||
|- | |- | ||
| | | | ||
| − | : | + | :The total sum is 166 carat. |
| + | :<math>\scriptstyle{\color{blue}{28+48+90=166}}</math> | ||
|style="text-align:right;"|עולי' קס"ו קראטי בין הכל | |style="text-align:right;"|עולי' קס"ו קראטי בין הכל | ||
|- | |- | ||
| | | | ||
| − | : | + | :Divide it by ten, as the number of ounces; each ounce is 16 carat and 3-fifths of one carat. |
| − | |style="text-align:right;"|חלקם | + | :<math>\scriptstyle{\color{blue}{\frac{28+48+90}{2+3+5}=\frac{166}{10}=16+\frac{3}{5}}}</math> |
| + | |style="text-align:right;"|חלקם בעשרות כמניין האוקיו‫'<br> | ||
יבא לכל אונקי' י"ו קראטי וג' חומשי קראטי | יבא לכל אונקי' י"ו קראטי וג' חומשי קראטי | ||
|- | |- | ||
| | | | ||
| + | :The same for any number you wish. | ||
|style="text-align:right;"|וכן לכל חשבון שתרצה | |style="text-align:right;"|וכן לכל חשבון שתרצה | ||
|- | |- | ||
| | | | ||
| − | === Pricing Problems === | + | === <span style=color:green>Pricing Problems</span> === |
| | | | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:gold|629|3Fmu}} | + | *{{#annot:gold|629|3Fmu}}When you know that the gold is 18 carat per ounce and you wish to know how many peraḥim is one ounce worth. |
:<math>\scriptstyle\frac{18}{3}</math> | :<math>\scriptstyle\frac{18}{3}</math> | ||
| − | |style="text-align:right;"|כשתדע שהזהב הוא מי"ח קראטי האונקי' ותרצה לדעת כמה פרחי' שוה האוקי‫'{{#annotend:3Fmu}} | + | |style="text-align:right;"|<big>כשתדע שהזהב הוא מי"ח קראטי האונקי'</big> ותרצה לדעת כמה פרחי' שוה האוקי‫'{{#annotend:3Fmu}} |
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\frac{18}{3}=6}}</math> | + | :Convert every 18 carat into thirds; 18 thirds are 6 integers. So, every ounce of gold that is 18 carat per ounce is worth 6 peraḥim. |
| + | :<math>\scriptstyle{\color{blue}{\frac{18}{3}=6}}</math> | ||
|style="text-align:right;"|עשה מכל י"ח קראטי שלישיות וי"ח שלישיות הם ו' שלמים<br> | |style="text-align:right;"|עשה מכל י"ח קראטי שלישיות וי"ח שלישיות הם ו' שלמים<br> | ||
הרי שהזהב שהוא מי"ח קראטי שוה האונקי' ממנו ו' פרחי‫' | הרי שהזהב שהוא מי"ח קראטי שוה האונקי' ממנו ו' פרחי‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | :But, you should subtract 6 pešiṭim for each carat, since the gold is not [pure?]. |
|style="text-align:right;"|אמת שאתה צרי' לפחות מכל קראטי ו' פשי' בעבור שזה הזהב אינו קוניינטו | |style="text-align:right;"|אמת שאתה צרי' לפחות מכל קראטי ו' פשי' בעבור שזה הזהב אינו קוניינטו | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:gold|629|T9hO}} | + | *{{#annot:gold|629|T9hO}}If the gold is 20 carat [per ounce] and you wish to know how much is one ounce worth? |
:<math>\scriptstyle\frac{20}{3}</math> | :<math>\scriptstyle\frac{20}{3}</math> | ||
| − | |style="text-align:right;"|ואם הזהב מעשרי' קראטי ותרצה לדע' כמה שוה האונקי‫'{{#annotend:T9hO}} | + | |style="text-align:right;"|<big>ואם הזהב</big> מעשרי' קראטי ותרצה לדע' כמה שוה האונקי‫'{{#annotend:T9hO}} |
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\frac{20}{3}=6+\frac{2}{3}}}</math> | + | :Convert the 20 carat into thirds. So, every ounce of gold that is 20 carat per ounce is worth 6 peraḥim and 2-thirds of one peraḥ. |
| + | :<math>\scriptstyle{\color{blue}{\frac{20}{3}=6+\frac{2}{3}}}</math> | ||
|style="text-align:right;"|עשה מן הכ' קראטי שלישיות<br> | |style="text-align:right;"|עשה מן הכ' קראטי שלישיות<br> | ||
הרי שהזהב שהוא מכ' קראטי שוה אונקי' ממנו ו' פרחי' וב' שלישי פרח | הרי שהזהב שהוא מכ' קראטי שוה אונקי' ממנו ו' פרחי' וב' שלישי פרח | ||
|- | |- | ||
| | | | ||
| − | : | + | :You should always subtract 6 pešiṭim for each carat. |
|style="text-align:right;"|ואתה צרי' לעולם לפחות ו"פ לכל קראטו | |style="text-align:right;"|ואתה צרי' לעולם לפחות ו"פ לכל קראטו | ||
|- | |- | ||
| | | | ||
| − | * | + | *If the gold is 21 carat [per ounce]. |
:<math>\scriptstyle\frac{21}{3}</math> | :<math>\scriptstyle\frac{21}{3}</math> | ||
|style="text-align:right;"|וכן אם הזהב הוא מכ"א קראטי | |style="text-align:right;"|וכן אם הזהב הוא מכ"א קראטי | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\frac{21}{3}=7}}</math> | + | :Say: 21 thirds are 7 integers; so the ounce is worth 7 peraḥim. |
| − | |style="text-align:right;"|אמור כ"א שלישיות הם ז' שלימים הרי ששוה | + | :<math>\scriptstyle{\color{blue}{\frac{21}{3}=7}}</math> |
| + | |style="text-align:right;"|אמור כ"א שלישיות הם ז' שלימים הרי <s>שבאוקי</s> ששוה <sup>ה</sup>אוקי' ז' פרחי‫' | ||
|- | |- | ||
| | | | ||
| − | * | + | *If the gold is 24 carat [per ounce] |
:<math>\scriptstyle\frac{24}{3}</math> | :<math>\scriptstyle\frac{24}{3}</math> | ||
|style="text-align:right;"|וכן אם הזהב הוא מכ"ד קראטי | |style="text-align:right;"|וכן אם הזהב הוא מכ"ד קראטי | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\frac{24}{3}=8}}</math> | + | :Say: 24 thirds are 8 integers; so the ounce is worth 8 peraḥim. |
| + | :<math>\scriptstyle{\color{blue}{\frac{24}{3}=8}}</math> | ||
|style="text-align:right;"|אמור כ"ד שלישיות הם ח' שלימים הרי שהאוקי' שוה ח' פרחי‫' | |style="text-align:right;"|אמור כ"ד שלישיות הם ח' שלימים הרי שהאוקי' שוה ח' פרחי‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | :You should always subtract 6 pešiṭim for each carat. |
|style="text-align:right;"|ואתה צריך לפחות ו' פשיטי לכל קראטו | |style="text-align:right;"|ואתה צריך לפחות ו' פשיטי לכל קראטו | ||
|- | |- | ||
| | | | ||
| − | : | + | :So, each ounce is worth 12 dinar. |
| − | |||
|style="text-align:right;"|שיבואו י"ב דינרי' בין כל האונקיו‫' | |style="text-align:right;"|שיבואו י"ב דינרי' בין כל האונקיו‫' | ||
|- | |- | ||
| | | | ||
| − | :: | + | :The ounce weighs 8 peraḥim that are 18 pešuṭim for each peraḥ. |
| − | |style="text-align:right;"|והאונקי' שוקלת ח' | + | :<math>\scriptstyle{\color{OliveGreen}{6\sdot24=12\sdot12=8\sdot18}}</math> |
| + | |style="text-align:right;"|והאונקי' שוקלת ח' פרחי' שיבואו <s>ד</s> י"ח [פשוטי']‫<ref>MS Ithaca פרחי' כ'</ref> לכל פרח | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|הרי שהזהב מפרח שאינו קוניינטו שוה י"ח | + | :So, the gold that is not [pure?] is worth 18 pešuṭim minus one peraḥ [?] |
| + | |style="text-align:right;"|הרי שהזהב מפרח שאינו קוניינטו שוה י"ח פ' פחו' מא' פרח | ||
|- | |- | ||
| | | | ||
| − | === Shared Work Problem === | + | === <span style=color:green>Shared Work Problem - Four rivers</span> === |
| | | | ||
| Line 3,676: | Line 4,833: | ||
:If all are flowing together, how long will it take them to fill [the fountain]? | :If all are flowing together, how long will it take them to fill [the fountain]? | ||
:<math>\scriptstyle X+\frac{1}{2}X+\frac{1}{3}X+\frac{1}{4}X=1</math> | :<math>\scriptstyle X+\frac{1}{2}X+\frac{1}{3}X+\frac{1}{4}X=1</math> | ||
| − | |style="text-align:right;"|ארבע נהרות רצים אל | + | |style="text-align:right;"|<big>ארבע נהרות רצים אל מעיין אחד</big><br> |
האחד ממלאו ביום אחד<br> | האחד ממלאו ביום אחד<br> | ||
והשני ממלאו בשני ימים<br> | והשני ממלאו בשני ימים<br> | ||
| Line 3,684: | Line 4,841: | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''False Position:'''</span> Do as follows: say: one, a half, a third, and a quarter are found in 12. | |
| + | :<math>\scriptstyle{\color{blue}{12}}</math> | ||
|style="text-align:right;"|עשה על זה הדרך אמור ראשון ושליש ורביע ימצאו י"ב | |style="text-align:right;"|עשה על זה הדרך אמור ראשון ושליש ורביע ימצאו י"ב | ||
|- | |- | ||
| | | | ||
| − | : | + | :One is 12. |
| − | |style="text-align:right;"|הראשון הוא י"ב | + | |style="text-align:right;"|הראשון הוא י"ב |
| − | |||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | :The half is 6. |
| + | |style="text-align:right;"|החצי ו‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | :The third is 4. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|השליש ד‫' |
|- | |- | ||
| | | | ||
| − | : | + | :The quarter is 3. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|הרביעי ג‫' |
|- | |- | ||
| | | | ||
| − | : | + | :<span style=color:green>'''Denominator:'''</span> Add them together; they are 25 and this is the denominator. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|חבר אותם יחד יהיו כ"ה והוא המחלק |
| + | |- | ||
| + | | colspan="2"| | ||
| + | :<math>\scriptstyle{\color{blue}{12+\left(\frac{1}{2}\sdot12\right)+\left(\frac{1}{3}\sdot12\right)+\left(\frac{1}{3}\sdot12\right)=12+6+4+3=25}}</math> | ||
|- | |- | ||
| | | | ||
| − | : | + | :When you look, you find that [together] they fill 25 fountains in 12 days: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכשתתבונן תמצא שי"ב ימים ימלאו כ"ה מעיינות |
|- | |- | ||
| | | | ||
| − | : | + | :The one that fills it in one day - fills 12 fountains in 12 days. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כיצד הממלאו ביום אחד ביום בי"ב ימים ימלא י"ב מעיינות |
|- | |- | ||
| | | | ||
| − | ::<span style=color: | + | :The one that fills it in 2 days - fills 6 fountains in 12 days. |
| + | |style="text-align:right;"|והממלאו בב' ימים <s>בכ"ד ימים</s> בי"ב ימים ימלא ו' מעיינות | ||
| + | |- | ||
| + | | | ||
| + | :The one that fills it in 3 days - fills 4 fountains in 12 days. | ||
| + | |style="text-align:right;"|והממלאו בג' ימים בי"ב ימים ימלא [ד']‫<ref>MS Ithaca ג'</ref> מעיינות | ||
| + | |- | ||
| + | | | ||
| + | :The one that fills it in 4 days - fills 3 fountains in 12 days. | ||
| + | |style="text-align:right;"|והממלאו בד' ימים בי"ב ימים ימלא ג' מעיינות | ||
| + | |- | ||
| + | | | ||
| + | :So, the four together fill 25 fountains in 12 days. | ||
| + | |style="text-align:right;"|הרי שארבעתם יחד ימלאו בי"ב ימים כ"ה מעיינות | ||
| + | |- | ||
| + | | | ||
| + | :<span style=color:green>'''Rule of Three:'''</span> Say: if 25 fountains are filled in 12 days, in how many parts of the day will it take for one fountain to be filled? | ||
| + | :<math>\scriptstyle{\color{blue}{25:12=1:X}}</math> | ||
|style="text-align:right;"|אמור אם כן כ"ה מעיינות יתמלאו בי"ב ימים א' מעיין בכמה חלק מן היום יתמלא | |style="text-align:right;"|אמור אם כן כ"ה מעיינות יתמלאו בי"ב ימים א' מעיין בכמה חלק מן היום יתמלא | ||
|- | |- | ||
| | | | ||
| − | ::together they fill | + | :Say: 1 time 12 is 12. Divide it by 25; the result is 12 parts of 25. So, when all four flow together, they fill it in 12 parts of 25 of a day. |
| − | |style="text-align:right;"|אמור א' פעם י"ב י"ב חלקם בכ"ה יבואו י"ב חלקים מכ"ה הרי שי"ב חלקים מכ"ה מהיום ממלאים אותו כשירוצו מארבעתם יחד | + | :<math>\scriptstyle{\color{blue}{X=\frac{1\sdot12}{25}=\frac{12}{25}}}</math> |
| + | |style="text-align:right;"|אמור א' פעם י"ב י"ב חלקם בכ"ה יבואו י"ב חלקים מכ"ה הרי שי"ב חלקים מכ"ה ‫<ref>40v</ref>מהיום ממלאים אותו כשירוצו מארבעתם יחד | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|ואם תרצה לדעת כמה חלק מן המים שם כל אחד מן הנהרות במעיין | + | :If you want to know how much of the water flows from each of the rivers to the fountain: |
| + | |style="text-align:right;"|<big>ואם תרצה לדעת</big> כמה חלק מן המים שם כל אחד מן הנהרות במעיין | ||
|- | |- | ||
| | | | ||
| − | : | + | :Say: the first is 12; 12 times 1, for the fountain, which is one, is 12. Divide it by 25; the result is 12 parts of 25. So, the one that fills it alone in 1 day, fills 12 parts of 25 in one day. |
| + | :<math>\scriptstyle{\color{blue}{X=\frac{1\sdot12}{25}=\frac{12}{25}}}</math> | ||
|style="text-align:right;"|אמור הראשון הוא י"ב וי"ב פעמים א' בעבור המעיין שהוא א' הרי י"ב<br> | |style="text-align:right;"|אמור הראשון הוא י"ב וי"ב פעמים א' בעבור המעיין שהוא א' הרי י"ב<br> | ||
| − | חלקם בכ"ה יבואו י"ב חלקים מכ"ה א"כ הממלאו ביום אחד שם בו ביום אחד י"ב חלקים מכ"ה | + | חלקם בכ"ה יבואו י"ב חלקים מכ"ה<br> |
| + | א"כ הממלאו ביום אחד שם בו ביום אחד י"ב חלקים מכ"ה | ||
|- | |- | ||
| | | | ||
| − | : | + | :For the one that fills it alone in 2 days, say: a half of 12 is 6. Say: 6 times 1, for the fountain, is 6. Divide it by 25; the result is 6 parts of 25. So, it fills 6 parts of 25 of the fountain. |
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{2}X=\frac{\frac{1}{2}\sdot12}{25}=\frac{6\sdot1}{25}=\frac{6}{25}}}</math> | ||
|style="text-align:right;"|ואותו הממלאו בב' ימים אמור החצי מי"ב הוא ו' אמור ו' פעמי' א' בעבור המעיין הם ו‫'<br> | |style="text-align:right;"|ואותו הממלאו בב' ימים אמור החצי מי"ב הוא ו' אמור ו' פעמי' א' בעבור המעיין הם ו‫'<br> | ||
חלקם בכ"ה יבואו ו' חלקים מכ"ה הרי ששם במעיין ו' חלקים מכ"ה | חלקם בכ"ה יבואו ו' חלקים מכ"ה הרי ששם במעיין ו' חלקים מכ"ה | ||
|- | |- | ||
| | | | ||
| − | : | + | :For the one that fills it alone in 3 days, say: a third of 12 is 4. Say: 4 times 1 is 4. Divide it by 25; the result is 4 parts of 25. |
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{3}X=\frac{\frac{1}{3}\sdot12}{25}=\frac{4\sdot1}{25}=\frac{4}{25}}}</math> | ||
|style="text-align:right;"|והממלאו בג' ימים אמור השליש מי"ב הוא ד' אמו' ד' פעמי' א' ד‫'<br> | |style="text-align:right;"|והממלאו בג' ימים אמור השליש מי"ב הוא ד' אמו' ד' פעמי' א' ד‫'<br> | ||
חלקם בכ"ה יבואו ד' חלקים מכ"ה | חלקם בכ"ה יבואו ד' חלקים מכ"ה | ||
|- | |- | ||
| | | | ||
| − | : | + | :For the one that fills it alone in 4 days, say: a quarter of 12 is 3. Say: 3 times 1 is 3. Divide it by 25; it fills 3 parts of 25 of the fountain. <math>\scriptstyle{\color{blue}{\frac{1}{4}X=\frac{\frac{1}{4}\sdot12}{25}=\frac{3\sdot1}{25}=\frac{3}{25}}}</math> |
|style="text-align:right;"|והממלאו בד' ימים אמור הרביע מי"ב הוא ג' אמור ג' פעמים א' ג‫'<br> | |style="text-align:right;"|והממלאו בד' ימים אמור הרביע מי"ב הוא ג' אמור ג' פעמים א' ג‫'<br> | ||
חלקם בכ"ה הרי ששם במעיין ג' חלקי' מכ"ה מן המים | חלקם בכ"ה הרי ששם במעיין ג' חלקי' מכ"ה מן המים | ||
|- | |- | ||
| | | | ||
| + | :Do this way for any number you wish. | ||
|style="text-align:right;"|ועל זה הדרך תעשה לכל חשבון שתרצה | |style="text-align:right;"|ועל זה הדרך תעשה לכל חשבון שתרצה | ||
|- | |- | ||
| | | | ||
| − | === Multiple Quantities Problems === | + | === <span style=color:green>Multiple Quantities Problems</span> === |
| | | | ||
| Line 3,763: | Line 4,948: | ||
:They went and took as he said and found out that they all received the same share equally. | :They went and took as he said and found out that they all received the same share equally. | ||
:How many were the sons, how many were the peraḥim, and how many peraḥim did each receive? | :How many were the sons, how many were the peraḥim, and how many peraḥim did each receive? | ||
| − | + | |style="text-align:right;"|<big>אדם אחד היה לו בנים לא נדע מספרם</big><br> | |
| − | |style="text-align:right;"|אדם אחד היה לו בנים לא נדע מספרם<br> | + | <big>והיו לו</big> פרחי' לא אומר לך כמה<br> |
| − | והיו לו פרחי' לא אומר לך כמה<br> | ||
אמר לראשון לך בארגז וקח א' פרח והעשירית מכל הנשאר<br> | אמר לראשון לך בארגז וקח א' פרח והעשירית מכל הנשאר<br> | ||
ולשיני אמר קח לך ב' פרחי' והעשירית מכל הנשאר<br> | ולשיני אמר קח לך ב' פרחי' והעשירית מכל הנשאר<br> | ||
וכן אמר לכל בניו<br> | וכן אמר לכל בניו<br> | ||
ולאחרון אמר קח כל מה שנשאר בארגז<br> | ולאחרון אמר קח כל מה שנשאר בארגז<br> | ||
| − | הלכו ולקחו כמו שאמר ומצאו שכלם קבלו בשוה<br> | + | הלכו ולקחו כמו שאמר ומצאו שקב שכלם קבלו בשוה<br> |
ועתה אמור כמה היו הבנים וכמה היו הפרחי' וכמה פרחי' באו לחלק כל אחד ואחד{{#annotend:WJGo}} | ועתה אמור כמה היו הבנים וכמה היו הפרחי' וכמה פרחי' באו לחלק כל אחד ואחד{{#annotend:WJGo}} | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | :<math>\scriptstyle1+\left[\frac{1}{10}\sdot\left(X-1\right)\right]=2+\frac{1}{10}\sdot\left[X-\left[\left[1+\left[\frac{1}{10}\sdot\left(X-1\right)\right]\right]+2\right]\right]</math> | ||
|- | |- | ||
| | | | ||
| − | ::the | + | :Do this way: since the fraction in this calculation is a tenth, take its analogous ten. Subtract one from it; 9 remains, so the sons are 9. |
| − | |style="text-align:right;"|עשה על זה הדרך בעבור שהחשבון | + | :<math>\scriptstyle{\color{blue}{10-\left(\frac{1}{10}\sdot10\right)=10-1=9}}</math> |
| − | קח דוגמתו עשרה ותפחות מהם אחד ישארו ט' וט' היו הבנים | + | |style="text-align:right;"|עשה על זה הדרך בעבור שהחשבון השבור שבזה החשבון הוא עשירית קח דוגמתו עשרה ותפחות מהם אחד ישארו ט' וט' היו הבנים |
|- | |- | ||
| | | | ||
| − | : | + | :9 times 9 is [the number of] the peraḥim, which are 81. |
| + | :<math>\scriptstyle{\color{blue}{9\sdot9=81}}</math> | ||
|style="text-align:right;"|וט' פעמים ט' היו הפרחים והם פ"א | |style="text-align:right;"|וט' פעמים ט' היו הפרחים והם פ"א | ||
|- | |- | ||
| | | | ||
| − | : | + | :The share of each is 9 peraḥim. |
|style="text-align:right;"|וט' פרחים היה חלק כל אחד ואחד | |style="text-align:right;"|וט' פרחים היה חלק כל אחד ואחד | ||
|- | |- | ||
| | | | ||
| − | : | + | :The first received one peraḥ and a tenth of the remainder, which is 8. So, he received 9 peraḥim. |
| + | :<math>\scriptstyle{\color{blue}{1+\left[\frac{1}{10}\sdot\left(81-1\right)\right]=1+8=9}}</math> | ||
|style="text-align:right;"|כיצד הראשון קבל א' פרח מן הפ"א ועשירית הנשאר שהוא ח' הרי שקיבל ט' פרחי‫' | |style="text-align:right;"|כיצד הראשון קבל א' פרח מן הפ"א ועשירית הנשאר שהוא ח' הרי שקיבל ט' פרחי‫' | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{81-9=72}}</math> | + | :72 [peraḥim] remain. |
| + | :<math>\scriptstyle{\color{blue}{81-9=72}}</math> | ||
|style="text-align:right;"|ונשארו ע"ב | |style="text-align:right;"|ונשארו ע"ב | ||
|- | |- | ||
| | | | ||
| − | : | + | :The second received 2 peraḥim from the 72 and a tenth of the remainder, which is 7. So, he received 9 peraḥim. |
| + | :<math>\scriptstyle{\color{blue}{2+\left[\frac{1}{10}\sdot\left(72-2\right)\right]=2+7=9}}</math> | ||
|style="text-align:right;"|השני קבל ב' פרחים מן הע"ב ועשירית הנשאר שהם ז' הרי שקיבל ט' פרחי‫' | |style="text-align:right;"|השני קבל ב' פרחים מן הע"ב ועשירית הנשאר שהם ז' הרי שקיבל ט' פרחי‫' | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{72-9=63}}</math> | + | :63 [peraḥim] remain. |
| + | :<math>\scriptstyle{\color{blue}{72-9=63}}</math> | ||
|style="text-align:right;"|ונשארו ס"ג | |style="text-align:right;"|ונשארו ס"ג | ||
|- | |- | ||
| | | | ||
| − | : | + | :The third received 3 peraḥim from the 63 and a tenth of the remainder, which is 6. So, he received 9 peraḥim. |
| + | :<math>\scriptstyle{\color{blue}{3+\left[\frac{1}{10}\sdot\left(63-3\right)\right]=3+6=9}}</math> | ||
|style="text-align:right;"|השלישי קיבל ג' פרחי' מן הס"ג ועשירית הנשאר שהם ו' הרי שקבל ט' פרחי‫' | |style="text-align:right;"|השלישי קיבל ג' פרחי' מן הס"ג ועשירית הנשאר שהם ו' הרי שקבל ט' פרחי‫' | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{63-9=54}}</math> | + | :54 [peraḥim] remain. |
| + | :<math>\scriptstyle{\color{blue}{63-9=54}}</math> | ||
|style="text-align:right;"|ונשארו נ"ד | |style="text-align:right;"|ונשארו נ"ד | ||
|- | |- | ||
| | | | ||
| − | : | + | :The fourth received 4 [peraḥim] from the 54 and a tenth of the remainder, which is 5. So, he received 9 peraḥim. |
| + | :<math>\scriptstyle{\color{blue}{4+\left[\frac{1}{10}\sdot\left(54-4\right)\right]=4+5=9}}</math> | ||
|style="text-align:right;"|הרביעי קבל ד' מן הנ"ד ועשירית הנשאר שהם ה' פרחי' הרי שקבל ט‫' | |style="text-align:right;"|הרביעי קבל ד' מן הנ"ד ועשירית הנשאר שהם ה' פרחי' הרי שקבל ט‫' | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{54-9=45}}</math> | + | :45 [peraḥim] remain. |
| + | :<math>\scriptstyle{\color{blue}{54-9=45}}</math> | ||
|style="text-align:right;"|ונשארו מ"ה | |style="text-align:right;"|ונשארו מ"ה | ||
|- | |- | ||
| | | | ||
| − | : | + | :The fifth received 5 peraḥim from the 45 and a tenth of the remainder, which is 4. So, he received 9 peraḥim. |
| + | :<math>\scriptstyle{\color{blue}{5+\left[\frac{1}{10}\sdot\left(45-5\right)\right]=5+4=9}}</math> | ||
|style="text-align:right;"|החמשי קבל ה' פרחי' מן המ"ה ועשירית מן הנשאר שהם ד' הרי שקיבל ט‫' | |style="text-align:right;"|החמשי קבל ה' פרחי' מן המ"ה ועשירית מן הנשאר שהם ד' הרי שקיבל ט‫' | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{45-9=36}}</math> | + | :36 [peraḥim] remain. |
| + | :<math>\scriptstyle{\color{blue}{45-9=36}}</math> | ||
|style="text-align:right;"|ונשארו ל"ו | |style="text-align:right;"|ונשארו ל"ו | ||
|- | |- | ||
| | | | ||
| − | : | + | :The sixth received 6 peraḥim from the 36 and a tenth of the remainder, which is 3. |
| − | |style="text-align:right;"|הששי קבל ו' פרחי' מן הל"ו ועשירית מן הנשאר שהם ג‫' | + | :<math>\scriptstyle{\color{blue}{6+\left[\frac{1}{10}\sdot\left(36-6\right)\right]=6+3=9}}</math> |
| + | |style="text-align:right;"|הששי קבל ו' פרחי' א מן הל"ו ועשירית מן הנשאר שהם ג‫' | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{36-9=27}}</math> | + | :27 [peraḥim] remain. |
| + | :<math>\scriptstyle{\color{blue}{36-9=27}}</math> | ||
|style="text-align:right;"|ונשארו כ"ז | |style="text-align:right;"|ונשארו כ"ז | ||
|- | |- | ||
| | | | ||
| − | : | + | :The seventh received 7 peraḥim from the 27 and a tenth of the remaining 20, which is 2. So, he received 9 peraḥim. |
| + | :<math>\scriptstyle{\color{blue}{7+\left[\frac{1}{10}\sdot\left(27-7\right)\right]=7+2=9}}</math> | ||
|style="text-align:right;"|השביעי קיבל ז' פרחי' מן הכ"ז ועשירית הכ' הנשארי' שהם ב' הרי שקבל ט' פרחי‫' | |style="text-align:right;"|השביעי קיבל ז' פרחי' מן הכ"ז ועשירית הכ' הנשארי' שהם ב' הרי שקבל ט' פרחי‫' | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{27-9=18}}</math> | + | :18 [peraḥim] remain. |
| + | :<math>\scriptstyle{\color{blue}{27-9=18}}</math> | ||
|style="text-align:right;"|ונשארו י"ח | |style="text-align:right;"|ונשארו י"ח | ||
|- | |- | ||
| | | | ||
| − | : | + | :The eighth received 8 peraḥim from the 18 and a tenth of the remaining 10, which is 1. So, he received 9 peraḥim. |
| + | :<math>\scriptstyle{\color{blue}{8+\left[\frac{1}{10}\sdot\left(18-8\right)\right]=8+1=9}}</math> | ||
|style="text-align:right;"|השמיני לקח ח' פר' מן הי"ח ועשירית הי' הנשארים שהוא א' הרי שקבל ט' פרחי‫' | |style="text-align:right;"|השמיני לקח ח' פר' מן הי"ח ועשירית הי' הנשארים שהוא א' הרי שקבל ט' פרחי‫' | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{18-9=9}}</math> | + | :9 [peraḥim] remain. |
| + | :<math>\scriptstyle{\color{blue}{18-9=9}}</math> | ||
|style="text-align:right;"|ונשארו ט‫' | |style="text-align:right;"|ונשארו ט‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | :The ninth, which is the last, went and took all, which is the remaining 9 peraḥim. |
|style="text-align:right;"|התשיעי שהוא האחרון הלך ולקח הכל שהם הט' פרחי' הנשארי‫' | |style="text-align:right;"|התשיעי שהוא האחרון הלך ולקח הכל שהם הט' פרחי' הנשארי‫' | ||
|- | |- | ||
| | | | ||
| − | :9 | + | :So, the sons are 9; the peraḥim are 9 times 9; and the share of each is 9 peraḥim. |
|style="text-align:right;"|הרי שט' היו הבני' וט' פע' ט' היו הפרחי' וט' פרחי' היה חלק כל אחד ואחד | |style="text-align:right;"|הרי שט' היו הבני' וט' פע' ט' היו הפרחי' וט' פרחי' היה חלק כל אחד ואחד | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|ודע כי זאת הריגולא היא כללם | + | :Know that this is a general rule. |
| + | |style="text-align:right;"|‫<ref>41r</ref><big>ודע כי זאת הריגולא</big> היא כללם | ||
|- | |- | ||
| | | | ||
| Line 3,866: | Line 5,071: | ||
:and so [he said] to all of them; | :and so [he said] to all of them; | ||
:and to the last [son] he said: take all that remained | :and to the last [son] he said: take all that remained | ||
| − | :<math>\scriptstyle1+\left[\frac{1}{9}\sdot\left(X-1\right)\right]=2+\frac{1}{9}\sdot\left[X\left[\left[1+\left[\frac{1}{9}\sdot\left(X-1\right)\right]\right]+2\right]\right]</math> | + | :<math>\scriptstyle1+\left[\frac{1}{9}\sdot\left(X-1\right)\right]=2+\frac{1}{9}\sdot\left[X-\left[\left[1+\left[\frac{1}{9}\sdot\left(X-1\right)\right]\right]+2\right]\right]</math> |
|style="text-align:right;"|וכמו כן אם אמר לראשון קח א' פרח ותשיעית הנשאר<br> | |style="text-align:right;"|וכמו כן אם אמר לראשון קח א' פרח ותשיעית הנשאר<br> | ||
ולשני ב' ותשיעית הנשאר<br> | ולשני ב' ותשיעית הנשאר<br> | ||
| Line 3,873: | Line 5,078: | ||
|- | |- | ||
| | | | ||
| − | : | + | :Do according to the way mentioned, i.e. since the fraction in this calculation is a ninth, take its analogous nine and subtract one from it; eight remains, so the sons are eight. |
| − | |style="text-align:right;"|יעשה על דרך הנזכר דהיינו בעבור שהחשבון השבור בזה החשבון הוא | + | :<math>\scriptstyle{\color{blue}{9-\left(\frac{1}{9}\sdot9\right)=9-1=8}}</math> |
| + | |style="text-align:right;"|יעשה על דרך הנזכר דהיינו בעבור שהחשבון השבור בזה החשבון הוא תש<sup>י</sup>עית<br> | ||
יקח דוגמתו תשעה ויפחות מהם אחד וישארו שמנה ושמנה היו הבנים | יקח דוגמתו תשעה ויפחות מהם אחד וישארו שמנה ושמנה היו הבנים | ||
|- | |- | ||
| | | | ||
| − | : | + | :8 times 8 are 64; so the peraḥim are 64. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{8\sdot8=64}}</math> |
| + | |style="text-align:right;"|וח' פע' ח' שהם ס"ד וס"ד היו הפרחים | ||
|- | |- | ||
| | | | ||
| − | : | + | :So, each received 8 peraḥim. |
|style="text-align:right;"|וח' פרחי' יבואו לכל אחד ואחד | |style="text-align:right;"|וח' פרחי' יבואו לכל אחד ואחד | ||
|- | |- | ||
| | | | ||
| + | :The same for any number you wish. | ||
|style="text-align:right;"|וכן לכל חשבון שתרצה | |style="text-align:right;"|וכן לכל חשבון שתרצה | ||
|- | |- | ||
| | | | ||
| − | === Rent Problems === | + | === <span style=color:green>Rent Problems</span> === |
| | | | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:a day|615|Jwr3}}If the house is rented at 6 liṭra a year or if you have [some money] that yields 6 liṭra a year, and you want to know how much it will yield a day | + | *{{#annot:a day|615|Jwr3}}If the house is rented at 6 liṭra a year or if you have [some money] that yields 6 liṭra a year, and you want to know how much it will yield a day: |
:<math>\scriptstyle\frac{6\sdot\left(20\sdot12\right)}{360}=\frac{X}{1}</math> | :<math>\scriptstyle\frac{6\sdot\left(20\sdot12\right)}{360}=\frac{X}{1}</math> | ||
| − | |style="text-align:right;"|אם יהיה שכירות הבית ו' ליטרין לשנה או אם יהיה לך חשבון שירויח השנה ו' ליטרי' ותרצה לידע כמה יבא היום{{#annotend:Jwr3}} | + | |style="text-align:right;"|<big>אם יהיה שכירות הבית ו' ליטרין</big> לשנה או אם יהיה לך חשבון שירויח השנה ו' ליטרי' ותרצה לידע כמה יבא היום{{#annotend:Jwr3}} |
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{X=\frac{2}{3}\sdot6=4}}</math> | + | :Take 2-thirds of 6, which is 4; so it yields 4 pešiṭim a day. |
| + | :<math>\scriptstyle{\color{blue}{X=\frac{2}{3}\sdot6=4}}</math> | ||
|style="text-align:right;"|תפוש הב' שלישיות מו' שהם ד' וכן יבואו אילו ד"פ ליום אחד | |style="text-align:right;"|תפוש הב' שלישיות מו' שהם ד' וכן יבואו אילו ד"פ ליום אחד | ||
|- | |- | ||
| Line 3,909: | Line 5,118: | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{X=\frac{2}{3}\sdot9=6}}</math> | + | :Take 2 parts of 9, which is 6; so it yields 6 pešiṭim a day. |
| + | :<math>\scriptstyle{\color{blue}{X=\frac{2}{3}\sdot9=6}}</math> | ||
|style="text-align:right;"|קח מב' חלקים מט' שהם ו' יבואו ו"פ ליום | |style="text-align:right;"|קח מב' חלקים מט' שהם ו' יבואו ו"פ ליום | ||
|- | |- | ||
| Line 3,918: | Line 5,128: | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{X=\frac{2}{3}\sdot12=8}}</math> | + | :Take two-thirds, which is 8; so it yields 8 pešiṭim a day. |
| + | :<math>\scriptstyle{\color{blue}{X=\frac{2}{3}\sdot12=8}}</math> | ||
|style="text-align:right;"|קח שני שלשיות והם ח' וכן יבואו ח' פשיטים בכל יום | |style="text-align:right;"|קח שני שלשיות והם ח' וכן יבואו ח' פשיטים בכל יום | ||
|- | |- | ||
| − | | | + | |Or, do this way: double the [number of] liṭra earned a year, then divide them into a third; the result are the pešiṭim earned a day. |
| − | :<math>\scriptstyle X=\frac{1}{3}\sdot2a</math> | + | :<math>\scriptstyle{\color{OliveGreen}{\frac{a\sdot\left(20\sdot12\right)}{360}=\frac{X}{1}}}</math> |
| − | |style="text-align:right;"|או תעשה בעניין זה תכפול הליטר' שיבואו השנה וחלקם לשליש וכמספר העולה יהיו | + | :<math>\scriptstyle{\color{OliveGreen}{X=\frac{1}{3}\sdot2a}}</math> |
| + | |style="text-align:right;"|או תעשה בעניין זה תכפול הליטר' שיבואו השנה וחלקם לשליש וכמספר העולה יהיו הפשיטי' שיבואו ליום | ||
|- | |- | ||
| | | | ||
| − | : | + | :Example: if is rented at 6 liṭra a year: |
| − | |style="text-align:right;"|דמיון זה אם יהיה השכירו ו' ליטרין השנה | + | |style="text-align:right;"|<big>דמיון זה</big> אם יהיה השכירו ו' ליטרין השנה |
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{X=\frac{2\sdot6}{3}=\frac{12}{3}=4}}</math> | + | :Double it; it is 12. Divide it into a third; it is 4; so it yields 4 pešiṭim a day. |
| + | :<math>\scriptstyle{\color{blue}{X=\frac{2\sdot6}{3}=\frac{12}{3}=4}}</math> | ||
|style="text-align:right;"|תכפלם יהיו י"ב חלקם לשליש יהיו ד' הרי שיבואו ד"פ ליום | |style="text-align:right;"|תכפלם יהיו י"ב חלקם לשליש יהיו ד' הרי שיבואו ד"פ ליום | ||
|- | |- | ||
| | | | ||
| − | : | + | :If is rented at 9 liṭra a year: |
|style="text-align:right;"|וכן אם יהיה השכירות ט' ליטרי' השנה | |style="text-align:right;"|וכן אם יהיה השכירות ט' ליטרי' השנה | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{X=\frac{2\sdot9}{3}=\frac{18}{3}=6}}</math> | + | :Double it; it is 18. Divide it into a third; it is 6; so it yields 6 pešiṭim a day. |
| + | :<math>\scriptstyle{\color{blue}{X=\frac{2\sdot9}{3}=\frac{18}{3}=6}}</math> | ||
|style="text-align:right;"|כפלם יהיו י"ח חלקם לשליש יהיו ו' הרי שיבואו ו' פשיטי' ליום | |style="text-align:right;"|כפלם יהיו י"ח חלקם לשליש יהיו ו' הרי שיבואו ו' פשיטי' ליום | ||
|- | |- | ||
| | | | ||
| − | : | + | :If is rented at 12 liṭra a year: |
|style="text-align:right;"|וכן אם יהיה השכירות י"ב ליט' השנה | |style="text-align:right;"|וכן אם יהיה השכירות י"ב ליט' השנה | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{X=\frac{2\sdot12}{3}=\frac{24}{3}=8}}</math> | + | :Double it; it is 24. Divide it into a third; it is 8; so [it yields] 8 pešiṭim a day. |
| − | |style="text-align:right;"|כפלם יהיו כ"ד חלקם לשליש יהיו ח' וכן יהיו ח"פ ליום | + | :<math>\scriptstyle{\color{blue}{X=\frac{2\sdot12}{3}=\frac{24}{3}=8}}</math> |
| + | |style="text-align:right;"|כפלם יהיו כ"ד חלקם לשליש יהיו [ח']‫<ref>MS Ithaca י"ח</ref> וכן יהיו ח"פ ליום | ||
|- | |- | ||
| | | | ||
| + | :Do this way for any similar calculation. | ||
|style="text-align:right;"|ועל זה הדרך תעשה לכל חשבון הדומה לזה | |style="text-align:right;"|ועל זה הדרך תעשה לכל חשבון הדומה לזה | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:a year|615|ZsTH}} | + | *{{#annot:a year|615|ZsTH}}If a man says: the house is rented at, or [the money] yields 4 pešiṭim a day, how many liṭra will be earned a year? |
:<math>\scriptstyle\frac{4}{1}=\frac{X\sdot\left(20\sdot12\right)}{360}</math> | :<math>\scriptstyle\frac{4}{1}=\frac{X\sdot\left(20\sdot12\right)}{360}</math> | ||
| − | |style="text-align:right;"|ואם יאמר אדם שכירות הבית או ריוח חשבון אחד עולה ד"פ ליום כמה ליט' יבואו השנה{{#annotend:ZsTH}} | + | |style="text-align:right;"|<big>ואם יאמר אדם [שכירות הבית או ריוח</big> חשבון אחד עולה ד"פ ליום כמה ליט' יבואו השנה{{#annotend:ZsTH}} |
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{X=4+\left(\frac{1}{2}\sdot4\right)=4+2=6}}</math> | + | :Add to 4 its half, which is 2; it is 6; so it yields at this rate 6 liṭra a year. |
| − | |style="text-align:right;"|הוסף על ד' חציים שהם ב' ויהיו ו' וכן יבואו לחשבון זה ו' ליטרי' השכיר' השנה | + | :<math>\scriptstyle{\color{blue}{X=4+\left(\frac{1}{2}\sdot4\right)=4+2=6}}</math> |
| + | |style="text-align:right;"|הוסף על ד' חציים שהם ב' ויהיו ו' וכן יבואו לחשבון זה ו' ליטרי' [השכיר']‫<ref>MS Ithaca om.</ref> השנה]‫<ref>MS Ithaca twice</ref> | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:a year|615|i7R1}} | + | *{{#annot:a year|615|i7R1}}If it is said: the house is rented at 6 pešiṭim a day, how many liṭra will be earned a year. |
:<math>\scriptstyle\frac{6}{1}=\frac{X\sdot\left(20\sdot12\right)}{360}</math> | :<math>\scriptstyle\frac{6}{1}=\frac{X\sdot\left(20\sdot12\right)}{360}</math> | ||
| − | |style="text-align:right;"|וכן אם יאמר שכירות הבית ו' פשיטי' ליום כמה ליטרי' יבואו השנה{{#annotend:i7R1}} | + | |style="text-align:right;"|‫<ref>41v</ref>וכן אם יאמר שכירות הבית ו' ליטרי' בשנה ו' פשיטי' ליום כמה ליטרי' יבואו השנה{{#annotend:i7R1}} |
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{X=6+\left(\frac{1}{2}\sdot6\right)=6+3=9}}</math> | + | :Add to 6 its half; it is 9; so it yields a year. |
| + | :<math>\scriptstyle{\color{blue}{X=6+\left(\frac{1}{2}\sdot6\right)=6+3=9}}</math> | ||
|style="text-align:right;"|הוסף על ו' חציים ג' הוא ט' וכן יבא ט' לשנה | |style="text-align:right;"|הוסף על ו' חציים ג' הוא ט' וכן יבא ט' לשנה | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:a year|615|m6t3}} | + | *{{#annot:a year|615|m6t3}}If it is said: the house is rented at 8 pešiṭim a day, how many liṭra will be earned a year |
:<math>\scriptstyle\frac{8}{1}=\frac{X\sdot\left(20\sdot12\right)}{360}</math> | :<math>\scriptstyle\frac{8}{1}=\frac{X\sdot\left(20\sdot12\right)}{360}</math> | ||
|style="text-align:right;"|וכן אם יאמר שכירות הבית הוא ח' פשיטים ליום כמה ליטרי' יבאו השנה{{#annotend:m6t3}} | |style="text-align:right;"|וכן אם יאמר שכירות הבית הוא ח' פשיטים ליום כמה ליטרי' יבאו השנה{{#annotend:m6t3}} | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{X=8+\left(\frac{1}{2}\sdot8\right)=8+4=12}}</math> | + | :Add to 8 its half, which is 4; it is 12; so it yields 12 liṭra a year. |
| − | |style="text-align:right;"|הוסף על ח' חציים שהם ד' יהיו י"ב וכך יהיו י"ב ליטרי' לשנ' ועל זה הדרך תעשה כל חשבון שתרצה | + | :<math>\scriptstyle{\color{blue}{X=8+\left(\frac{1}{2}\sdot8\right)=8+4=12}}</math> |
| + | |style="text-align:right;"|הוסף על ח' חציים שהם ד' יהיו י"ב וכך יהיו י"ב ליטרי' לשנ‫' | ||
| + | |- | ||
| + | | | ||
| + | :Do this way for any number you want. | ||
| + | |style="text-align:right;"|ועל זה הדרך תעשה כל חשבון שתרצה | ||
|- | |- | ||
| | | | ||
| − | === Pricing Problems === | + | === <span style=color:green>Pricing Problems</span> === |
| | | | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:liṭra|629|vMs9}}If you buy one kikkar for 7 liṭra, and you want to know how much one liṭra is worth | + | *{{#annot:liṭra|629|vMs9}}If you buy one kikkar for 7 liṭra, and you want to know how much one liṭra is worth. |
:<math>\scriptstyle\frac{7\sdot\left(20\sdot12\right)}{100}=\frac{X}{1}</math> | :<math>\scriptstyle\frac{7\sdot\left(20\sdot12\right)}{100}=\frac{X}{1}</math> | ||
| − | |style="text-align:right;"|אם תקנה הככר מן הלוחות או מאיזה דבר שתרצה בז' ליט' ותרצה לדעת כמה יבא האחד{{#annotend:vMs9}} | + | |style="text-align:right;"|‫<ref>MS Ithaca om.</ref>אם תקנה הככר מן הלוחות או מאיזה דבר שתרצה בז' ליט' ותרצה לדעת כמה יבא האחד{{#annotend:vMs9}} |
|- | |- | ||
| | | | ||
| − | : | + | :Take 2 pešuṭim and 2-fifths for each liṭra. |
|style="text-align:right;"|קח לכל ליט' ב' פשוטי' וב' חמישייו‫' | |style="text-align:right;"|קח לכל ליט' ב' פשוטי' וב' חמישייו‫' | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{X=7\sdot\left(2+\frac{2}{5}\right)=14+\frac{14}{5}=17-\frac{1}{5}}}</math> | + | :Then, double 7; they are 14 pešuṭim and 14-fifths; so the total is 17 pešuṭim minus a fifth. |
| + | :<math>\scriptstyle{\color{blue}{X=7\sdot\left(2+\frac{2}{5}\right)=14+\frac{14}{5}=17-\frac{1}{5}}}</math> | ||
|style="text-align:right;"|אם כן תכפול ז' ויהיו י"ד פשוטי' וי"ד חומשים הרי בן כולם י"ז פשוטי' פחו' מחומש | |style="text-align:right;"|אם כן תכפול ז' ויהיו י"ד פשוטי' וי"ד חומשים הרי בן כולם י"ז פשוטי' פחו' מחומש | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:liṭra|629|wqjc}}If you buy one kikkar for 5 liṭra | + | *{{#annot:liṭra|629|wqjc}}If you buy one kikkar for 5 liṭra. |
:<math>\scriptstyle\frac{5\sdot\left(20\sdot12\right)}{100}=\frac{X}{1}</math> | :<math>\scriptstyle\frac{5\sdot\left(20\sdot12\right)}{100}=\frac{X}{1}</math> | ||
|style="text-align:right;"|וכן אם תקנה הככר בה' ליט‫'{{#annotend:wqjc}} | |style="text-align:right;"|וכן אם תקנה הככר בה' ליט‫'{{#annotend:wqjc}} | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{X=5\sdot\left(2+\frac{2}{5}\right)=10+\frac{10}{5}=12}}</math> | + | :Double 5; it is 10. So, at this rate it is worth 10 pešuṭim and 10-fifths that are 12 pešuṭim. |
| + | :<math>\scriptstyle{\color{blue}{X=5\sdot\left(2+\frac{2}{5}\right)=10+\frac{10}{5}=12}}</math> | ||
|style="text-align:right;"|כפול ה' יהיו י' הרי שיבוא לזה החשבו' י' פשוטי' וי' חמשייו' שהם י"ב פשוטים | |style="text-align:right;"|כפול ה' יהיו י' הרי שיבוא לזה החשבו' י' פשוטי' וי' חמשייו' שהם י"ב פשוטים | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:liṭra|629|UbD9}}If you buy one kikkar for 9 liṭra | + | *{{#annot:liṭra|629|UbD9}}If you buy one kikkar for 9 liṭra. |
:<math>\scriptstyle\frac{9\sdot\left(20\sdot12\right)}{100}=\frac{X}{1}</math> | :<math>\scriptstyle\frac{9\sdot\left(20\sdot12\right)}{100}=\frac{X}{1}</math> | ||
|style="text-align:right;"|וכן אם תקנה הככר בט' ליט' ליט‫'{{#annotend:UbD9}} | |style="text-align:right;"|וכן אם תקנה הככר בט' ליט' ליט‫'{{#annotend:UbD9}} | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{X=9\sdot\left(2+\frac{2}{5}\right)=18+\frac{18}{5}=21+\frac{3}{5}}}</math> | + | :Double 9; it is 18 and 18-fifths, which are 21 pešuṭim and 3-fifths. |
| + | :<math>\scriptstyle{\color{blue}{X=9\sdot\left(2+\frac{2}{5}\right)=18+\frac{18}{5}=21+\frac{3}{5}}}</math> | ||
|style="text-align:right;"|כפול ט' יהיו יהיו י"ח וי"ח חמשיות שהם כ"א פשו' וג' חמשיות | |style="text-align:right;"|כפול ט' יהיו יהיו י"ח וי"ח חמשיות שהם כ"א פשו' וג' חמשיות | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:liṭra|629|Hnv6}}One kikkar for 25 liṭra | + | *{{#annot:liṭra|629|Hnv6}}One kikkar for 25 liṭra. |
:<math>\scriptstyle\frac{25\sdot\left(20\sdot12\right)}{100}=\frac{X}{1}</math> | :<math>\scriptstyle\frac{25\sdot\left(20\sdot12\right)}{100}=\frac{X}{1}</math> | ||
|style="text-align:right;"|וכן לחשבון כ"ה ליט' הככר{{#annotend:Hnv6}} | |style="text-align:right;"|וכן לחשבון כ"ה ליט' הככר{{#annotend:Hnv6}} | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{X=25\sdot\left(2+\frac{2}{5}\right)=50+\frac{50}{5} | + | :One is worth 50 pešuṭim and 50-fifths, which are 5 [dinar]. |
| − | + | :<math>\scriptstyle{\color{blue}{X=25\sdot\left(2+\frac{2}{5}\right)=50+\frac{50}{5}=5\sdot12}}</math> | |
|style="text-align:right;"|יבא האחד נ' פשו' ונ' חמשיות שהם ה‫' | |style="text-align:right;"|יבא האחד נ' פשו' ונ' חמשיות שהם ה‫' | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:liṭra|629|8VD0}}One kikkar for 30 liṭra | + | *{{#annot:liṭra|629|8VD0}}One kikkar for 30 liṭra. |
:<math>\scriptstyle\frac{30\sdot\left(20\sdot12\right)}{100}=\frac{X}{1}</math> | :<math>\scriptstyle\frac{30\sdot\left(20\sdot12\right)}{100}=\frac{X}{1}</math> | ||
|style="text-align:right;"|וכן לחשבון ל' ליט' הככר{{#annotend:8VD0}} | |style="text-align:right;"|וכן לחשבון ל' ליט' הככר{{#annotend:8VD0}} | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{X=30\sdot\left(2+\frac{2}{5}\right)=60+\frac{60}{5} | + | :One is worth 60 pešuṭim and 60-fifths, which are 6 dinar. |
| − | + | :<math>\scriptstyle{\color{blue}{X=30\sdot\left(2+\frac{2}{5}\right)=60+\frac{60}{5}=6\sdot12}}</math> | |
|style="text-align:right;"|יבא האחד ס' פשו' וס' חמשיות שהם ו' דינ‫' | |style="text-align:right;"|יבא האחד ס' פשו' וס' חמשיות שהם ו' דינ‫' | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:liṭra|629|zA6t}} | + | *{{#annot:liṭra|629|zA6t}}If there are dinar included, for example: one kikkar for 10 liṭra and 3 dinar. |
:<math>\scriptstyle\frac{\left[10\sdot\left(20\sdot12\right)\right]+\left(3\sdot12\right)}{100}=\frac{X}{1}</math> | :<math>\scriptstyle\frac{\left[10\sdot\left(20\sdot12\right)\right]+\left(3\sdot12\right)}{100}=\frac{X}{1}</math> | ||
|style="text-align:right;"|ואם יהיו שם די' כגון שיבא הככר י' ליט' וג' די‫'{{#annotend:zA6t}} | |style="text-align:right;"|ואם יהיו שם די' כגון שיבא הככר י' ליט' וג' די‫'{{#annotend:zA6t}} | ||
|- | |- | ||
| | | | ||
| − | : | + | :Take for each dinar 3 parts of 25 [of one pašuṭ]. |
|style="text-align:right;"|קח לכל די' ג' חלקים מכ"ה | |style="text-align:right;"|קח לכל די' ג' חלקים מכ"ה | ||
|- | |- | ||
| | | | ||
| − | : | + | :The 10 liṭra are worth 2 dinar, as you know. |
| + | :<math>\scriptstyle{\color{blue}{10\sdot\left(2+\frac{2}{5}\right)=2\sdot12}}</math> | ||
|style="text-align:right;"|ומן הי' ליט' יבואו ב' די' כמו שידעת | |style="text-align:right;"|ומן הי' ליט' יבואו ב' די' כמו שידעת | ||
|- | |- | ||
| | | | ||
| − | : | + | :The 3 dinar are worth 9 parts of 25. |
| + | :<math>\scriptstyle{\color{blue}{\frac{3}{25}\sdot3=\frac{9}{25}}}</math> | ||
|style="text-align:right;"|ובעבו' הג' די' יבואו ט' חלקים מכ"ה | |style="text-align:right;"|ובעבו' הג' די' יבואו ט' חלקים מכ"ה | ||
|- | |- | ||
| | | | ||
| + | :So, always add 3 parts of 25 for each dinar. | ||
|style="text-align:right;"|וכן לעולם תוסיף לכל די' ג' חלקים מכ"ה | |style="text-align:right;"|וכן לעולם תוסיף לכל די' ג' חלקים מכ"ה | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | === Interest and Discount Problems - Find the Earned Interest === | + | === <span style=color:green>Interest and Discount Problems - Find the Earned Interest</span> === |
| | | | ||
| Line 4,068: | Line 5,295: | ||
:You want to know: how many pešuṭim for one liṭra a month should be given? | :You want to know: how many pešuṭim for one liṭra a month should be given? | ||
:<math>\scriptstyle\frac{12\sdot\left(20\sdot12\right)}{12\sdot100}=\frac{X}{1\sdot1}</math> | :<math>\scriptstyle\frac{12\sdot\left(20\sdot12\right)}{12\sdot100}=\frac{X}{1\sdot1}</math> | ||
| − | |style="text-align:right;"|ואם תתן י"ב ליט' מן הככר לשנה<br> | + | |style="text-align:right;"|‫[ואם תתן י"ב ליט' מן הככר לשנה<br> |
ותרצה לידע לחשבו' כמה פשוטי' הליט' יבוא החודש{{#annotend:19vp}} | ותרצה לידע לחשבו' כמה פשוטי' הליט' יבוא החודש{{#annotend:19vp}} | ||
|- | |- | ||
| | | | ||
| − | : | + | :Take one fifth [of a pašuṭ] for each liṭra given for the kikkar. |
|style="text-align:right;"|תפוש מכל ליט' שיבא הככר א' חומש | |style="text-align:right;"|תפוש מכל ליט' שיבא הככר א' חומש | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{X=\frac{1}{5}\sdot12=\frac{12}{5}=2+\frac{2}{5}}}</math> | + | :So, one liṭra a month is worth 12-fifths, i.e. 2 pešuṭim and 2-fifths. |
| + | :<math>\scriptstyle{\color{blue}{X=\frac{1}{5}\sdot12=\frac{12}{5}=2+\frac{2}{5}}}</math> | ||
|style="text-align:right;"|אם כן יבא לחשבו' י"ב חומשי' דהיינו לחשבו' ב' פשוטי' וב' חמישייו' החודש הליט‫' | |style="text-align:right;"|אם כן יבא לחשבו' י"ב חומשי' דהיינו לחשבו' ב' פשוטי' וב' חמישייו' החודש הליט‫' | ||
|- | |- | ||
| Line 4,085: | Line 5,313: | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{X=\frac{1}{5}\sdot7=\frac{7}{5}=1+\frac{2}{5}}}</math> | + | :It is worth 7-fifths, which is 1 pašuṭ and 2-fifths. |
| + | :<math>\scriptstyle{\color{blue}{X=\frac{1}{5}\sdot7=\frac{7}{5}=1+\frac{2}{5}}}</math> | ||
|style="text-align:right;"|לחשבו' ז' חומשים שהוא א' פשוט וב' חמישייו‫' | |style="text-align:right;"|לחשבו' ז' חומשים שהוא א' פשוט וב' חמישייו‫' | ||
|- | |- | ||
| | | | ||
| − | + | *{{#annot:for one liṭra a month|638|J7xa}}At 8 liṭra for one kikkar a year. | |
| − | + | :<math>\scriptstyle\frac{8\sdot\left(20\sdot12\right)}{12\sdot100}=\frac{X}{1\sdot1}</math> | |
|style="text-align:right;"|ולחשבון ח' ליט' הככר לשנה{{#annotend:J7xa}} | |style="text-align:right;"|ולחשבון ח' ליט' הככר לשנה{{#annotend:J7xa}} | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{X=\frac{1}{5}\sdot8=1+\frac{3}{5}}}</math> | + | :One liṭra a month is worth [8]-fifths, which is 1 pašuṭ and 3-fifths of [one pašuṭ]. |
| + | :<math>\scriptstyle{\color{blue}{X=\frac{1}{5}\sdot8=1+\frac{3}{5}}}</math> | ||
|style="text-align:right;"|יבא חמשים שהם א' פשוט וג' חמשי' פשוטי' לליט' בחדש | |style="text-align:right;"|יבא חמשים שהם א' פשוט וג' חמשי' פשוטי' לליט' בחדש | ||
|- | |- | ||
| | | | ||
| − | + | *{{#annot:for one liṭra a month|638|aRVG}}At 10 liṭra for one kikkar a year. | |
| − | + | :<math>\scriptstyle\frac{10\sdot\left(20\sdot12\right)}{12\sdot100}=\frac{X}{1\sdot1}</math> | |
|style="text-align:right;"|ולחש[ב]ון י' ליט' הככר לשנה{{#annotend:aRVG}} | |style="text-align:right;"|ולחש[ב]ון י' ליט' הככר לשנה{{#annotend:aRVG}} | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{X=\frac{1}{5}\sdot10=\frac{10}{5}=2}}</math> | + | :One liṭra a month is worth 10-fifths, which is 2 pešuṭim. |
| + | :<math>\scriptstyle{\color{blue}{X=\frac{1}{5}\sdot10=\frac{10}{5}=2}}</math> | ||
|style="text-align:right;"|יבא לחשבון י' חמשים שהוא לחשבון ב' פשו' החדש לליט‫' | |style="text-align:right;"|יבא לחשבון י' חמשים שהוא לחשבון ב' פשו' החדש לליט‫' | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"|וכן לכל חשבון שתרצה | + | :The same for any number you want. |
| + | |style="text-align:right;"|וכן לכל חשבון שתרצה]‫<ref>MS Ithaca om.</ref> | ||
|- | |- | ||
| | | | ||
| Line 4,113: | Line 5,345: | ||
:You want to know: how much will be earned in one month? | :You want to know: how much will be earned in one month? | ||
:<math>\scriptstyle\frac{6}{1\sdot20}=\frac{X}{1\sdot3}</math> | :<math>\scriptstyle\frac{6}{1\sdot20}=\frac{X}{1\sdot3}</math> | ||
| − | |style="text-align:right;"|אם תלוה שלשה דינרי' לחשבון ו' פשיטי' החדש הליטר‫'<br> | + | |style="text-align:right;"|<big>אם תלוה שלשה דינרי' לחשבון ו' פשיטי'</big> החדש הליטר‫'<br> |
ותרצה לידע כמה יבא החדש{{#annotend:nf4P}} | ותרצה לידע כמה יבא החדש{{#annotend:nf4P}} | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> Say: if 20 dinar yield 6 pešiṭim, how much will 3 dinar yield? | |
| − | |style="text-align:right;"|אמור אם מכ' דינרים יבא ו' פשיטי' מג' דינרי כמה יעלו יבאו | + | :<math>\scriptstyle{\color{blue}{20:6=3:X}}</math> |
| + | |style="text-align:right;"|אמור אם מכ' דינרים יבא ו' פשיטי' מג' דינרי' כמה יעלו יבאו | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{X=\frac{3\sdot6}{20}=\frac{18}{20}}}</math> | + | :Say: 3 times 6 is 18. Divide it by 20; the result is 18 parts of 20 of one pašuṭ. |
| + | :<math>\scriptstyle{\color{blue}{X=\frac{3\sdot6}{20}=\frac{18}{20}}}</math> | ||
|style="text-align:right;"|אמור ג"פ ו' י"ח<br> | |style="text-align:right;"|אמור ג"פ ו' י"ח<br> | ||
חלקם בכ' יבואו י"ח חלקים מכ' בפשוט | חלקם בכ' יבואו י"ח חלקים מכ' בפשוט | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:for some dinar a month|638|601f}}You lend 5 dinar at 7 pešuṭim for one liṭra a | + | *{{#annot:for some dinar a month|638|601f}}You lend 5 dinar at 7 pešuṭim for one liṭra a year. |
:You want to know: how much will be earned in one month? | :You want to know: how much will be earned in one month? | ||
:<math>\scriptstyle\frac{7}{1\sdot20}=\frac{X}{1\sdot5}</math> | :<math>\scriptstyle\frac{7}{1\sdot20}=\frac{X}{1\sdot5}</math> | ||
| − | |style="text-align:right;"|וכן אם תלוה ה' דינרי' לחשבון ז' פשיטי' | + | |style="text-align:right;"|‫[וכן אם תלוה ה' דינרי' לחשבון]‫<ref>MS Ithaca twice</ref> ז' פשיטי' הלי' השנה<br> |
ותרצה לידע כמה יבא בחדש{{#annotend:601f}} | ותרצה לידע כמה יבא בחדש{{#annotend:601f}} | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> Say: if 20 dinar yield 7 pešiṭim, how much will 5 dinar yield? | |
| + | :<math>\scriptstyle{\color{blue}{20:7=5:X}}</math> | ||
|style="text-align:right;"|אמור אם מכ' דינרי' יבא ז' פשי' מה' דינרי' כמה יבואו | |style="text-align:right;"|אמור אם מכ' דינרי' יבא ז' פשי' מה' דינרי' כמה יבואו | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{X=\frac{5\sdot7}{20}=\frac{35}{20}=1+\frac{15}{20}}}</math> | + | :Say: 5 times 7 is 35. Divide it by 20; the result is 1 pašuṭ and 15 parts of 20 of one pašuṭ. |
| + | :<math>\scriptstyle{\color{blue}{X=\frac{5\sdot7}{20}=\frac{35}{20}=1+\frac{15}{20}}}</math> | ||
|style="text-align:right;"|אמור ה' פעמים ז' ל"ה<br> | |style="text-align:right;"|אמור ה' פעמים ז' ל"ה<br> | ||
חלקם בכ' יבואו א' פשוט וט"ו חלקים מכ' בפשוט | חלקם בכ' יבואו א' פשוט וט"ו חלקים מכ' בפשוט | ||
|- | |- | ||
| | | | ||
| + | :Always apply this way. | ||
|style="text-align:right;"|ועל זה הדרך תעשה לעולם | |style="text-align:right;"|ועל זה הדרך תעשה לעולם | ||
|- | |- | ||
| Line 4,148: | Line 5,385: | ||
:You want to know: how much will be earned? | :You want to know: how much will be earned? | ||
:<math>\scriptstyle\frac{6}{1\sdot20}=\frac{X}{9\sdot3}</math> | :<math>\scriptstyle\frac{6}{1\sdot20}=\frac{X}{9\sdot3}</math> | ||
| − | |style="text-align:right;"|אם תלוה שלשה דינרי' לחשבון ו' פשו' הליט' בחדש ועמדו ט' חדשי' ותרצה לידע כמה יבואו{{#annotend:RXKm}} | + | |style="text-align:right;"|<big>אם תלוה שלשה דינרי' לחשבון [ו' פשו' הליט' בחדש]</big>‫<ref>MS Ithaca ופה"ה</ref> ועמדו ט' חדשי' ותרצה לידע כמה יבואו{{#annotend:RXKm}} |
|- | |- | ||
| | | | ||
| − | :: | + | :Say: 3 times 9 is 27. So, 3 dinar yield in 9 month as 27 dinar yield in one month at 6 pešuṭim for one liṭra a year. |
| − | |style="text-align:right;"|אמור ג' פעמים ט' הם כ"ז<br> | + | :<math>\scriptstyle{\color{blue}{3\sdot9=27}}</math> |
| − | וכמו שיבואו כ"ז דינרי' א' חודש לחשבון ו' | + | |style="text-align:right;"|אמור ג' פעמים [ט']‫<ref>MS Ithaca om.</ref> הם כ"ז<br> |
| + | וכמו שיבואו כ"ז דינרי' א' חודש לחשבון ול ו"פ הלי' השנה כן יבאו ג' דינרי' ט' חדשים | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:for some dinar, some months|638|5I0F}}You lend 7 dinar for 10 months at 5 pešuṭim for one liṭra a | + | *{{#annot:for some dinar, some months|638|5I0F}}You lend 7 dinar for 10 months at 5 pešuṭim for one liṭra a year. |
:You want to know: how much will be earned? | :You want to know: how much will be earned? | ||
:<math>\scriptstyle\frac{5}{1\sdot20}=\frac{X}{10\sdot7}</math> | :<math>\scriptstyle\frac{5}{1\sdot20}=\frac{X}{10\sdot7}</math> | ||
| − | |style="text-align:right;"|וכן אם תלוה ז' דינרי' לחשבון ה' פשיטי' | + | |style="text-align:right;"|וכן אם תלוה ט ז' דינרי' לחשבון ה' פשיטי' הלי' השנה ועמדו יד י' חדשים ותרצה לדעת כמה יבאו{{#annotend:5I0F}} |
|- | |- | ||
| | | | ||
| − | :: | + | :Say: 7 times 10 is 70. So, 7 dinar yield in 10 month as 70 dinar that are 3 liṭra and a half yield in one month at 5 pešuṭim for one liṭra a year. |
| − | : | + | :<math>\scriptstyle{\color{blue}{7\sdot10=70=\left(3+\frac{1}{2}\right)\sdot20}}</math> |
|style="text-align:right;"|אמור ז' פעמי' י' הם ע‫'<br> | |style="text-align:right;"|אמור ז' פעמי' י' הם ע‫'<br> | ||
| − | וכמו שיבאו ע' דינרי' שהם ג' ליטרין וחצי א' חדש הליט' לחשבון ה' | + | וכמו שיבאו ע' דינרי' שהם ג' ליטרין וחצי א' חדש הליט' לחשבון ה' פשיטי' הליט' השנה כן יבואו הז' דינרי' י' חדשים |
|- | |- | ||
| | | | ||
| − | *{{#annot:for some dinar, some months|638|E3cW}}You lend 9 dinar for 5 months at 5 pešuṭim for one liṭra a | + | *{{#annot:for some dinar, some months|638|E3cW}}You lend 9 dinar for 5 months at 5 pešuṭim for one liṭra a year. |
:You want to know: how much will be earned? | :You want to know: how much will be earned? | ||
:<math>\scriptstyle\frac{5}{1\sdot20}=\frac{X}{5\sdot9}</math> | :<math>\scriptstyle\frac{5}{1\sdot20}=\frac{X}{5\sdot9}</math> | ||
| − | |style="text-align:right;"|וכן אם תלוה ט' דינרי' לחשבון ה' פשי' הליט' | + | |style="text-align:right;"|וכן אם תלוה ט' דינרי' לחשבון ה' פשי' הליט' השנה ועמדו ה' חדשים ותרצה לדעת כמה יבואו{{#annotend:E3cW}} |
|- | |- | ||
| | | | ||
| − | :: | + | :Say: 5 times 9 is 45. So, 9 dinar yield in 5 month as 45 dinar yield in one month at [5] pešuṭim for one liṭra a year. |
| + | :<math>\scriptstyle{\color{blue}{5\sdot9=45}}</math> | ||
|style="text-align:right;"|אמור ה' פעמי' ט' מ"ה<br> | |style="text-align:right;"|אמור ה' פעמי' ט' מ"ה<br> | ||
| − | וכמו שיבואו מ"ה דינרי' א' חדש לחשבון | + | וכמו שיבואו מ"ה דינרי' א' חדש לחשבון ד"פ הלי' השנה כן יבואו הט' דינרי' ה' חדשים |
|- | |- | ||
| | | | ||
| + | :The same for any number you wish. | ||
|style="text-align:right;"|וכן לכל חשבון שתרצה | |style="text-align:right;"|וכן לכל חשבון שתרצה | ||
|- | |- | ||
| Line 4,185: | Line 5,425: | ||
:You want to know: how much it will yield a month? | :You want to know: how much it will yield a month? | ||
:<math>\scriptstyle\frac{12\sdot20}{12\sdot1}=\frac{X}{1\sdot1}</math> | :<math>\scriptstyle\frac{12\sdot20}{12\sdot1}=\frac{X}{1\sdot1}</math> | ||
| − | |style="text-align:right;"|אם תלוה הככר בי"ב ליטרי' השנה<br> | + | |style="text-align:right;"|<big>אם תלוה הככר בי"ב ליטרי'</big> ‫<ref>42r</ref>השנה<br> |
ותרצה לידע כמה יבא החדש{{#annotend:w6vz}} | ותרצה לידע כמה יבא החדש{{#annotend:w6vz}} | ||
|- | |- | ||
| | | | ||
| − | : | + | :The rule: always multiply the [number of] liṭra earned in one year bu 5 and the result is the yadot. |
|style="text-align:right;"|דרך ריגולא תרבה הליט' שיבואו השנה לעולם על ה' ומה שיעלה יהיה ידות | |style="text-align:right;"|דרך ריגולא תרבה הליט' שיבואו השנה לעולם על ה' ומה שיעלה יהיה ידות | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{12\sdot5=60 | + | :For example, say: 12 times 5 is 60. We find that they are 60 yadot that are 20 dinar. |
| − | + | :<math>\scriptstyle{\color{blue}{12\sdot5=60=20\sdot3}}</math> | |
| − | |style="text-align:right;"|דמיון זה אמור י"ב פעמים ה' הם ס' נמצא שהם ס' ידות<br> | + | |style="text-align:right;"|<big>דמיון זה</big> אמור י"ב פעמים ה' הם ס' נמצא שהם ס' ידות<br> |
שהם כ' דינרי‫' | שהם כ' דינרי‫' | ||
|- | |- | ||
| Line 4,206: | Line 5,446: | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{5\sdot5=25 | + | :Say: 5 times 5 is 25, which is 25 yadot that are 8 dinar and 4 pešiṭim; and so it yields a month. |
| − | + | :<math>\scriptstyle{\color{blue}{5\sdot5=25=\left(8+\frac{4}{12}\right)\sdot3}}</math> | |
| − | |style="text-align:right;"|אמור ה' פעמים ה' כ"ה והם כ"ה ידות | + | |style="text-align:right;"|אמור ה' פעמים [ה']‫<ref>MS Ithaca om.</ref> כ"ה והם כ"ה ידות שהם ח' דינרי' וד' פשיטי' וכן יבא החדש |
| − | שהם ח' דינרי' וד' פשיטי' וכן יבא החדש | ||
|- | |- | ||
| | | | ||
| Line 4,217: | Line 5,456: | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{5\sdot6=30 | + | :Say: 5 times 6 is 30, which is 30 yadot that are 10 dinar; and so it yields a month. |
| − | + | :<math>\scriptstyle{\color{blue}{5\sdot6=30=10\sdot3}}</math> | |
| − | |style="text-align:right;"|אמור ה' פעמים ו' הם ל' והם ל' ידות | + | |style="text-align:right;"|אמור ה' פעמים ו' הם ל' והם ל' ידות שהם י' דינרי' וכן יבא החדש |
| − | שהם י' דינרי' וכן יבא החדש | ||
|- | |- | ||
| | | | ||
| Line 4,228: | Line 5,466: | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{5\sdot8=40 | + | :Say: 5 times 8 is 40, which is 40 yadot that are 13 dinar and 4 pešiṭim; and so it yields a month. |
| − | + | :<math>\scriptstyle{\color{blue}{5\sdot8=40=\left(13+\frac{4}{12}\right)\sdot3}}</math> | |
| − | |style="text-align:right;"|אמור ה' פעמי' ח' הם מ' והם מ' ידות | + | |style="text-align:right;"|אמור ה' פעמי' ח' הם מ' והם מ' ידות שהם י"ג דינרי' וד"פ |
| − | שהם י"ג דינרי' וד"פ | ||
|- | |- | ||
| | | | ||
| + | :If there are dinar involved, take 1 pašuṭ for every dinar. | ||
|style="text-align:right;"|ואם יהיו שם דינרי' תפוש מכל דינר א' פשוט | |style="text-align:right;"|ואם יהיו שם דינרי' תפוש מכל דינר א' פשוט | ||
|- | |- | ||
| | | | ||
| + | :The same for any number you want. | ||
|style="text-align:right;"|וכן לכל חשבון שתרצה | |style="text-align:right;"|וכן לכל חשבון שתרצה | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | === Interest and Discount Problems (MS Verona) === | + | === <span style=color:green>Interest and Discount Problems (MS Verona)</span> === |
| | | | ||
| Line 4,251: | Line 5,488: | ||
:What remained was lent for another year and some of the money was cashed as before. | :What remained was lent for another year and some of the money was cashed as before. | ||
:Then what remained was lent for a third year at 4 pešuṭim for one liṭra a month. | :Then what remained was lent for a third year at 4 pešuṭim for one liṭra a month. | ||
| − | :At the end of the year some money was cashed as was done at the end of the first and the second year and no pašuṭ was left | + | :At the end of the year some money was cashed as was done at the end of the first and the second year and no pašuṭ was left. |
| − | |||
|style="text-align:right;"|הלוויתי כ' דינ' לחשבון ד' פשו' לליט' בחדש עמדו שנה אחת והחזיר קצת מהמעות<br> | |style="text-align:right;"|הלוויתי כ' דינ' לחשבון ד' פשו' לליט' בחדש עמדו שנה אחת והחזיר קצת מהמעות<br> | ||
והנשארים עמדו שנה אחת והחזיר קצת מהמעו' כמו שנתן בראשונה<br> | והנשארים עמדו שנה אחת והחזיר קצת מהמעו' כמו שנתן בראשונה<br> | ||
והנשארים עמדו שנה שלשית לחשבון ד' פשוטי' לליט' בחדש<br> | והנשארים עמדו שנה שלשית לחשבון ד' פשוטי' לליט' בחדש<br> | ||
ובסוף השנה נתן כל כך מעות כמו שנתן בסוף שנה ראשונה ושנייה ולא נשאר לתת שום פשוט{{#annotend:zwBr}} | ובסוף השנה נתן כל כך מעות כמו שנתן בסוף שנה ראשונה ושנייה ולא נשאר לתת שום פשוט{{#annotend:zwBr}} | ||
| − | |} | + | |- |
| − | { | + | | colspan="2"| |
| + | :<math>\scriptstyle20+4-X+\left[\frac{4}{20}\sdot\left(20+4-X\right)\right]-X+\left[\frac{4}{20}\sdot\left[20+4-X+\left[\frac{4}{20}\sdot\left(20+4-X\right)\right]-X\right]\right]=X</math> | ||
|- | |- | ||
| | | | ||
| − | : | + | :The three payments are 28 dinar, 5 pešuṭim and 73 parts of 91. |
| − | + | :<math>\scriptstyle{\color{blue}{3X=28+\frac{5+\frac{73}{91}}{12}}}</math> | |
|style="text-align:right;"|עולים הג' פרעונות כ"ח דינ' וה' פשוטים וע"ג חלקים מצ"א | |style="text-align:right;"|עולים הג' פרעונות כ"ח דינ' וה' פשוטים וע"ג חלקים מצ"א | ||
|- | |- | ||
| | | | ||
| − | : | + | :So, each payment is 9 dinar, 5 pešuṭim and 85 parts of 91. |
| − | + | :<math>\scriptstyle{\color{blue}{X=\frac{28+\frac{5+\frac{73}{91}}{12}}{3}=9+\frac{5+\frac{85}{91}}{12}}}</math> | |
|style="text-align:right;"|הרי שיבא בכל פרעון ופרעון ט' די' וה' פשו' ופ"ה חלקים מצ"א | |style="text-align:right;"|הרי שיבא בכל פרעון ופרעון ט' די' וה' פשו' ופ"ה חלקים מצ"א | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:for some liṭra, some months|638|Csxb}}I lent one liṭra for 3 pešuṭim a month. | + | *{{#annot:for some liṭra, some months|638|Csxb}}If a man asks you: I lent one liṭra for 3 pešuṭim a month. |
| − | :How | + | :How much will 60 liṭra make in 8 months? |
:<math>\scriptstyle\frac{3}{1\sdot1}=\frac{X\sdot\left(20\sdot12\right)}{8\sdot60}</math> | :<math>\scriptstyle\frac{3}{1\sdot1}=\frac{X\sdot\left(20\sdot12\right)}{8\sdot60}</math> | ||
|style="text-align:right;"|אם ישאלך אדם הלוותי מעות לחשבון ג' פשוטי' לליט' בחדש<br> | |style="text-align:right;"|אם ישאלך אדם הלוותי מעות לחשבון ג' פשוטי' לליט' בחדש<br> | ||
| Line 4,278: | Line 5,515: | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{3\sdot8=24}}</math> | + | :Multiply the 3 pešuṭim by the months and say: 3 times 8 is 24 pešuṭim that are 2 dinar. |
| + | :<math>\scriptstyle{\color{blue}{3\sdot8=24}}</math> | ||
|style="text-align:right;"|תרבה אלו הג' פשו' עם החדשים ואמור ג' פעמי' ח' הם כ"ד כ"ד פשוטי' שהם ב' דינ‫' | |style="text-align:right;"|תרבה אלו הג' פשו' עם החדשים ואמור ג' פעמי' ח' הם כ"ד כ"ד פשוטי' שהם ב' דינ‫' | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{2\sdot60=120 | + | :Then, multiply the 2 dinar by the 60 liṭra and say: 60 times 2 are 120 dinar that are 6 liṭra. So, 60 liṭra at one liṭra for 3 pešuṭim a month yield 6 liṭra in 8 months. |
| − | + | :<math>\scriptstyle{\color{blue}{2\sdot60=120=20\sdot6}}</math> | |
|style="text-align:right;"|אחר כך תרבה אלו הב' די' עם הס' ליט' ואמור ס' פעמים ב' הם ק"כ דינ' שהם ו' ליט‫'<br> | |style="text-align:right;"|אחר כך תרבה אלו הב' די' עם הס' ליט' ואמור ס' פעמים ב' הם ק"כ דינ' שהם ו' ליט‫'<br> | ||
נמצא הס' ליט' לחשבון ג' פשוטי' הליט' לחדש יבואו ו' ליט' לח' חדשים | נמצא הס' ליט' לחשבון ג' פשוטי' הליט' לחדש יבואו ו' ליט' לח' חדשים | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:liṭra for one day|666|L2vB}}I lent one liṭra for so and so pešuṭim a month. | + | *{{#annot:liṭra for one day|666|L2vB}}If a man says: I lent one liṭra for so and so pešuṭim a month. |
:How many liṭra will yield one pašuṭ a day? | :How many liṭra will yield one pašuṭ a day? | ||
:<math>\scriptstyle\frac{a}{30\sdot1}=\frac{1}{1\sdot X}</math> | :<math>\scriptstyle\frac{a}{30\sdot1}=\frac{1}{1\sdot X}</math> | ||
| Line 4,295: | Line 5,533: | ||
|- | |- | ||
| | | | ||
| − | : | + | :We divide 30 liṭra by as much money that one liṭra yields a month. |
| − | + | :<math>\scriptstyle{\color{blue}{X=\frac{30}{a}}}</math> | |
|style="text-align:right;"|נחלק ל' ליט' בכל כך חלקים כמו המעו' שהרויח הליט' לחדש | |style="text-align:right;"|נחלק ל' ליט' בכל כך חלקים כמו המעו' שהרויח הליט' לחדש | ||
|- | |- | ||
| | | | ||
| − | + | *{{#annot:liṭra for one day|666|3oVI}}Example: I lent one liṭra for 2 pešuṭim and a half a month. | |
| − | : | + | :You want to know how many liṭra will yield one pašuṭ a day at this price. |
| − | + | :<math>\scriptstyle\frac{2+\frac{1}{2}}{30\sdot1}=\frac{1}{1\sdot X}</math> | |
|style="text-align:right;"|המשל בזה הרי שהלוותי הליט' בב' פשו' וחצי לחדש<br> | |style="text-align:right;"|המשל בזה הרי שהלוותי הליט' בב' פשו' וחצי לחדש<br> | ||
ותרצה לידע כמה ליט' ירוויחו ביום אחד א' פשו' לפי זה החשבון{{#annotend:3oVI}} | ותרצה לידע כמה ליט' ירוויחו ביום אחד א' פשו' לפי זה החשבון{{#annotend:3oVI}} | ||
|- | |- | ||
| | | | ||
| − | : | + | :We divide 30 liṭra by 2 and a half; the result is 12 liṭra. We find that 12 liṭra yield one pašuṭ a day. |
| + | :<math>\scriptstyle{\color{blue}{X=\frac{30}{2+\frac{1}{2}}=12}}</math> | ||
|style="text-align:right;"|נחלק ל' ליט' בב' וחצי יבואו י"ב ליט‫'<br> | |style="text-align:right;"|נחלק ל' ליט' בב' וחצי יבואו י"ב ליט‫'<br> | ||
נמצא שבי"ב ליט' יבואו ביום אחד א' פשוט ביום אחד א' פשוט | נמצא שבי"ב ליט' יבואו ביום אחד א' פשוט ביום אחד א' פשוט | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:for some liṭra|660|YoJf}} | + | *{{#annot:for some liṭra|660|YoJf}}If a man says: one kikkar yields 37 liṭra, 18 dinar, and 7 pešuṭim a year. |
:How long will it take 65 liṭra to yield the same? | :How long will it take 65 liṭra to yield the same? | ||
:<math>\scriptstyle\frac{\left[37\sdot\left(20\sdot12\right)\right]+\left(18\sdot12\right)+7}{12\sdot100}=\frac{\left[37\sdot\left(20\sdot12\right)\right]+\left(18\sdot12\right)+7}{X\sdot65}</math> | :<math>\scriptstyle\frac{\left[37\sdot\left(20\sdot12\right)\right]+\left(18\sdot12\right)+7}{12\sdot100}=\frac{\left[37\sdot\left(20\sdot12\right)\right]+\left(18\sdot12\right)+7}{X\sdot65}</math> | ||
| Line 4,319: | Line 5,558: | ||
|- | |- | ||
| | | | ||
| − | :: | + | :Multiply the 100 liṭra by the 12 months of the year and say: 12 times 100 is 1200. |
| − | + | |style="text-align:right;"|תרבה הק' ליט' עם י"ב חדשי השנה ואמור י"ב פעמים ק' הם אלף ור‫' | |
| − | |style="text-align:right;"|תרבה הק' ליט' עם י"ב חדשי השנה ואמור י"ב פעמים ק' הם אלף ור‫' | + | |- |
| − | חלקם על ס"ה יבואו לכל חלק י" | + | | |
| + | :Divide it by 65; the quotient is [18] months, 13 days and 11 parts of 13 of one day, i.e. parts of 65 and this is the time it takes 65 liṭra to yield as much as the kikkar yields a year. | ||
| + | |style="text-align:right;"|חלקם על ס"ה יבואו לכל חלק י"ב חדשים וי"ג ימים וי"א חלקים מי"ג ביום ר"ל חלקים מס"ה ובזה הזמן ירוויחו ס"ה ליט' כמו שירויח הככר לשנה | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | :<math>\scriptstyle{\color{blue}{X=\frac{100\sdot12}{65}=\frac{1200}{65}=18+\frac{13+\frac{55}{65}}{30}=18+\frac{13+\frac{11}{13}}{30}}}</math> | ||
|- | |- | ||
| | | | ||
| − | : | + | :Whether the kikkar yields 37 liṭra, 18 dinar, and 7 pešuṭim a year as we [said], or 20 liṭra, or 30, or any number it may be. |
|style="text-align:right;"|בין לחשבון ל"ז ליט' וי"ח דינ' וז' פשו' הככר לשנה כמו שעשינו<br> | |style="text-align:right;"|בין לחשבון ל"ז ליט' וי"ח דינ' וז' פשו' הככר לשנה כמו שעשינו<br> | ||
בין לחשבון כ' ליט' בין לחשבון כ"ה בין לחשבון ל' בין לאי זה חשבון שיהיה | בין לחשבון כ' ליט' בין לחשבון כ"ה בין לחשבון ל' בין לאי זה חשבון שיהיה | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:for some liṭra|660|npGv}}20 liṭra yield 4 liṭra a year. | + | *{{#annot:for some liṭra|660|npGv}}If it is said: 20 liṭra yield 4 liṭra a year. |
:How long will it take 30 liṭra to yield the same? | :How long will it take 30 liṭra to yield the same? | ||
:<math>\scriptstyle\frac{4}{20\sdot12}=\frac{4}{X\sdot30}</math> | :<math>\scriptstyle\frac{4}{20\sdot12}=\frac{4}{X\sdot30}</math> | ||
| Line 4,337: | Line 5,581: | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{X=\frac{20\sdot12}{30}=\frac{240}{30}=8}}</math> | + | :Do as you did above and say: 20 times 12 is 240. Divide it into 30 parts; the result is 8 months. We find that 30 liṭra yield in 8 months the same as 20 liṭra yield in one year. |
| + | :<math>\scriptstyle{\color{blue}{X=\frac{20\sdot12}{30}=\frac{240}{30}=8}}</math> | ||
|style="text-align:right;"|עשה כמו שעשית למעלה ואמור כ' פעמים י"ב הם ר"מ<br> | |style="text-align:right;"|עשה כמו שעשית למעלה ואמור כ' פעמים י"ב הם ר"מ<br> | ||
חלקם בל' חלקים יבואו ח' חדשים<br> | חלקם בל' חלקים יבואו ח' חדשים<br> | ||
| Line 4,343: | Line 5,588: | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:days, for one liṭra|660|8P3l}}I lent one liṭra for 3 pešuṭim a month. | + | *{{#annot:days, for one liṭra|660|8P3l}}If it is said: I lent one liṭra for 3 pešuṭim a month. |
| − | : | + | :You want to know in how many days will one liṭra yield one pašuṭ. |
:<math>\scriptstyle\frac{3}{30\sdot1}=\frac{1}{X\sdot1}</math> | :<math>\scriptstyle\frac{3}{30\sdot1}=\frac{1}{X\sdot1}</math> | ||
|style="text-align:right;"|אם יאמר הלוויתי הליט' לחשבון ג' ג' פשו' הליט' בחדש<br> | |style="text-align:right;"|אם יאמר הלוויתי הליט' לחשבון ג' ג' פשו' הליט' בחדש<br> | ||
| Line 4,350: | Line 5,595: | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\frac{30}{3}=10}}</math> | + | :Divide 30 days into 3 parts; the result is 10 days for each part. So, one liṭra yields in 10 days 1 pašuṭ at 3 pešuṭim a month for one liṭra. |
| + | :<math>\scriptstyle{\color{blue}{\frac{30}{3}=10}}</math> | ||
|style="text-align:right;"|תחלק ל' יום בג' חלקים יבואו י' ימים לכל חלק<br> | |style="text-align:right;"|תחלק ל' יום בג' חלקים יבואו י' ימים לכל חלק<br> | ||
הרי שבי' ימים תרויח הליט' א' פשו' לחשבון ג' פשו' הליט' לחדש | הרי שבי' ימים תרויח הליט' א' פשו' לחשבון ג' פשו' הליט' לחדש | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> This calculation is as follows, say: if 3 pešuṭim are earned a day for one liṭra, in how many days will 1 pašuṭ be earned? | |
| + | :<math>\scriptstyle{\color{blue}{3:30=1:X}}</math> | ||
|style="text-align:right;"|וזה החשבון יוצא כך אמור אם ג' פשו' ירויחו לא' ליט' בא' יום א' פשוט בכמה ימים ירויח | |style="text-align:right;"|וזה החשבון יוצא כך אמור אם ג' פשו' ירויחו לא' ליט' בא' יום א' פשוט בכמה ימים ירויח | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{X=\frac{30\sdot1}{3}=\frac{30}{3}=10}}</math> | + | :Say: 1 time 30 is 30. Divide it by 3; the result is 10 days. |
| + | :<math>\scriptstyle{\color{blue}{X=\frac{30\sdot1}{3}=\frac{30}{3}=10}}</math> | ||
|style="text-align:right;"|אמור א' פעם ל' הוא ל‫'<br> | |style="text-align:right;"|אמור א' פעם ל' הוא ל‫'<br> | ||
חלקם בג' יבואו י' ימים | חלקם בג' יבואו י' ימים | ||
| Line 4,370: | Line 5,618: | ||
|- | |- | ||
| | | | ||
| − | : | + | :Divide 150 into as many parts as the [number of] liṭra earned by one kikkar a year. |
| − | + | :<math>\scriptstyle{\color{blue}{X=\frac{150}{a}}}</math> | |
|style="text-align:right;"|חלק ק"נ בכל כך חלקים כמו הליט' שירויח הככר לשנה | |style="text-align:right;"|חלק ק"נ בכל כך חלקים כמו הליט' שירויח הככר לשנה | ||
|- | |- | ||
| | | | ||
| − | + | *{{#annot:liṭra for one day|666|maiV}}Example: one kikkar yields 12 liṭra a year. | |
| − | : | + | :You wish to know how many liṭra will yield one pašuṭ a day? |
| − | + | :<math>\scriptstyle\frac{12\sdot\left(20\sdot12\right)}{360\sdot100}=\frac{1}{1\sdot X}</math> | |
|style="text-align:right;"|המשל בזה הככר ירויח י"ב ליט' בשנה<br> | |style="text-align:right;"|המשל בזה הככר ירויח י"ב ליט' בשנה<br> | ||
ותרצה לידע כמה ליט' ירויחו ביום אחד א' פשוט{{#annotend:maiV}} | ותרצה לידע כמה ליט' ירויחו ביום אחד א' פשוט{{#annotend:maiV}} | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{X=\frac{150}{12}=12+\frac{1}{2}}}</math> | + | :Divide 150 liṭra into 12 parts; the result is 12 liṭra and a half in each part. So, 12 liṭra and a half yield one pašuṭ a day. |
| + | :<math>\scriptstyle{\color{blue}{X=\frac{150}{12}=12+\frac{1}{2}}}</math> | ||
|style="text-align:right;"|חלק ק"נ ליט' בי"ב חלקים ויעלו י"ב ליט' וחצי בכל חלק<br> | |style="text-align:right;"|חלק ק"נ ליט' בי"ב חלקים ויעלו י"ב ליט' וחצי בכל חלק<br> | ||
| − | הרי שי"ב ליט' וחצי ירויחו ביום אחד א' פשוט וכן לכל חשבון כזה | + | הרי שי"ב ליט' וחצי ירויחו ביום אחד א' פשוט |
| + | |- | ||
| + | | | ||
| + | :The same for any similar calculation. | ||
| + | |style="text-align:right;"|וכן לכל חשבון כזה | ||
|- | |- | ||
| | | | ||
| Line 4,394: | Line 5,647: | ||
|- | |- | ||
| | | | ||
| − | : | + | :Divide 150 days into as many parts as the [number of] liṭra earned by one kikkar a year. |
| − | + | :<math>\scriptstyle{\color{blue}{X=\frac{150}{a}}}</math> | |
|style="text-align:right;"|חלק ק"נ ימים בכל כך חלקים כמו הליט' שירויח הככר לשנה | |style="text-align:right;"|חלק ק"נ ימים בכל כך חלקים כמו הליט' שירויח הככר לשנה | ||
|- | |- | ||
| | | | ||
| − | + | *{{#annot:days, for one liṭra|660|XbFW}}Example: one kikkar yields 9 liṭra a year. | |
| − | : | + | :You wish to know in how many days will one liṭra yield one pašuṭ? |
| − | + | :<math>\scriptstyle\frac{9\sdot\left(20\sdot12\right)}{360\sdot100}=\frac{1}{X\sdot1}</math> | |
|style="text-align:right;"|המשל בזה הככר ירויח ט' ליט' השנה<br> | |style="text-align:right;"|המשל בזה הככר ירויח ט' ליט' השנה<br> | ||
ותרצה לידע בכמה ימים תרויח הליט' א' פשו‫'{{#annotend:XbFW}} | ותרצה לידע בכמה ימים תרויח הליט' א' פשו‫'{{#annotend:XbFW}} | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{X=\frac{150}{9}=16+\frac{2}{3}}}</math> | + | :Divide 150 days into 9 parts; they are 16 days and 2-thirds of a day. So, one liṭra yields [one] pašuṭ in 16 days and 2-thirds of a day. |
| + | :<math>\scriptstyle{\color{blue}{X=\frac{150}{9}=16+\frac{2}{3}}}</math> | ||
|style="text-align:right;"|חלק ק"נ ימים בט' חלקים שהם י"ו ימים וב' שלישי יום<br> | |style="text-align:right;"|חלק ק"נ ימים בט' חלקים שהם י"ו ימים וב' שלישי יום<br> | ||
הרי שבי"ו ימים וב' שלישי יום תרויח הליט' ט' פשוט | הרי שבי"ו ימים וב' שלישי יום תרויח הליט' ט' פשוט | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:liṭra for some months|666|JBNu}} | + | *{{#annot:liṭra for some months|666|JBNu}}If a man says: so and so liṭra yield so and so liṭra in so and so [months]. |
:How many liṭra will yield the same in so and so [months]? | :How many liṭra will yield the same in so and so [months]? | ||
:<math>\scriptstyle\frac{a}{c\sdot b}=\frac{a}{d\sdot X}</math> | :<math>\scriptstyle\frac{a}{c\sdot b}=\frac{a}{d\sdot X}</math> | ||
| Line 4,418: | Line 5,672: | ||
|- | |- | ||
| | | | ||
| − | : | + | :Multiply the number of the months by the number of the liṭra, then divide them into as many parts as the [number of] months we wish to know. |
| − | + | :<math>\scriptstyle{\color{blue}{X=\frac{c\sdot b}{d}}}</math> | |
|style="text-align:right;"|תרבה סכום החדשי' כנגד סכום הליט' וחלקם בכך וכך חלקים כמו החדשי' שנרצה לידע | |style="text-align:right;"|תרבה סכום החדשי' כנגד סכום הליט' וחלקם בכך וכך חלקים כמו החדשי' שנרצה לידע | ||
|- | |- | ||
| | | | ||
| − | :*{{#annot:liṭra for some months|666|f21Z}}25 liṭra yield 40 dinar in 6 months. | + | :*{{#annot:liṭra for some months|666|f21Z}}Example: 25 liṭra yield 40 dinar in 6 months. |
::How many liṭra will yield the same in 8 months? | ::How many liṭra will yield the same in 8 months? | ||
::<math>\scriptstyle\frac{40}{6\sdot25}=\frac{40}{8\sdot X}</math> | ::<math>\scriptstyle\frac{40}{6\sdot25}=\frac{40}{8\sdot X}</math> | ||
| Line 4,430: | Line 5,684: | ||
|- | |- | ||
| | | | ||
| + | ::Multiply the number of the months by the number of the liṭra and say: 6 times 25, the result is 150. | ||
| + | |style="text-align:right;"|תרבה סכום החדשים כנגד סכום הליט' ואמור ו' פעמי' כ"ה יבוא ק"נ | ||
| + | |- | ||
| + | | | ||
| + | ::Divide it by 8 months; the result is 18 liṭra and 15 dinar. | ||
::<math>\scriptstyle{\color{blue}{X=\frac{6\sdot25}{8}=\frac{150}{8}=18+\frac{15}{20}}}</math> | ::<math>\scriptstyle{\color{blue}{X=\frac{6\sdot25}{8}=\frac{150}{8}=18+\frac{15}{20}}}</math> | ||
| − | + | |style="text-align:right;"|חלקם בח' חדשים יבואו י"ח ליט' וט"ו די‫' | |
| − | |style="text-align:right;"| | + | |- |
| − | חלקם בח' חדשים יבואו י"ח ליט' וט"ו די‫' | + | | |
| − | נמצא שי"ח ליט' וט"ו די' ירויחו בח' חדשים מ' די' כמו שירויח כ"ה ליטרין בו' חדשים | + | ::We find that 18 liṭra and 15 dinar yield in 8 months 40 dinar, as 25 liṭra yield in 6 months. |
| + | |style="text-align:right;"|נמצא שי"ח ליט' וט"ו די' ירויחו בח' חדשים מ' די' כמו שירויח כ"ה ליטרין בו' חדשים | ||
|- | |- | ||
| | | | ||
*{{#annot:years, for some liṭra|660|2pIQ}}I lent one liṭra for so and so [pešuṭim] a month. | *{{#annot:years, for some liṭra|660|2pIQ}}I lent one liṭra for so and so [pešuṭim] a month. | ||
| − | :In how many [years] will so and so liṭra be doubled? | + | :In how many [years] will so and so liṭra be doubled? |
:<math>\scriptstyle\frac{a}{1\sdot1}=\frac{\left(20\sdot12\right)\sdot b}{12\sdot X\sdot b}</math> | :<math>\scriptstyle\frac{a}{1\sdot1}=\frac{\left(20\sdot12\right)\sdot b}{12\sdot X\sdot b}</math> | ||
|style="text-align:right;"|אם יאמר אדם הלוויתי הליטרא בכך וכך לחדש כל כך<br> | |style="text-align:right;"|אם יאמר אדם הלוויתי הליטרא בכך וכך לחדש כל כך<br> | ||
| Line 4,444: | Line 5,704: | ||
|- | |- | ||
| | | | ||
| − | : | + | :Divide 20 years into as many parts as the [number of pešuṭim] that one liṭra yields a month. |
| − | + | :<math>\scriptstyle{\color{blue}{X=\frac{20}{a}}}</math> | |
|style="text-align:right;"|חלק כ' שנים בכל כך חלקים כמו המעות שתרוויח הליט' בחדש | |style="text-align:right;"|חלק כ' שנים בכל כך חלקים כמו המעות שתרוויח הליט' בחדש | ||
|- | |- | ||
| Line 4,455: | Line 5,715: | ||
|- | |- | ||
| | | | ||
| + | ::Divide 20 years into 3 parts; the result is that the 95 liṭra are doubled in 6 years and 8 months. | ||
::<math>\scriptstyle{\color{blue}{X=\frac{20}{3}=6+\frac{8}{12}}}</math> | ::<math>\scriptstyle{\color{blue}{X=\frac{20}{3}=6+\frac{8}{12}}}</math> | ||
| − | |||
|style="text-align:right;"|חלק כ' שנים בג' חלקים ויבא שבו' שנים וח' חדשים יכפלו הצ"ה ליט‫' | |style="text-align:right;"|חלק כ' שנים בג' חלקים ויבא שבו' שנים וח' חדשים יכפלו הצ"ה ליט‫' | ||
|- | |- | ||
| | | | ||
| − | ::Check: <math>\scriptstyle{\color{blue}{3\sdot\left(6+\frac{8}{12}\right)=3\sdot\left(6+\frac{2}{3}\right)=18+2=20}}</math> | + | ::<span style=color:green>'''Check:'''</span> This calculation is as follows: since the liṭra is for 3 pešuṭim a month, it yields 3 dinar a year. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וזה החשבון יוצא כך אחרי שהליט' היא בג' פשוטי' לחדש אם כן מרווחו בשנה ג' די‫' |
| − | כי בו' שנים יבואו י"ח די' ובח' חדשים ב' די' הרי כ' דינ‫' | + | |- |
| − | וכמו שיכפלו כ' די' בו' שנים וח' חדשים כן בזה הזמן יכפלו הצ"ה ליט‫' | + | | |
| − | ולכן אמר חלק | + | ::The 3 dinar are multiplied by 6 years and 2-thirds of a year: by 6 years; the result is 18 dinar; by 8 months; it is 2 dinar; so they are 20 dinar. |
| + | ::<math>\scriptstyle{\color{blue}{3\sdot\left(6+\frac{8}{12}\right)=3\sdot\left(6+\frac{2}{3}\right)=18+2=20}}</math> | ||
| + | |style="text-align:right;"|ויכפלו הג' די' בו' שנים וב' שלישי שנה כי בו' שנים יבואו י"ח די' ובח' חדשים ב' די' הרי כ' דינ‫' | ||
| + | |- | ||
| + | | | ||
| + | ::As 20 dinar are doubled in 6 years and 8 months, so the 95 liṭra are doubled in that time. | ||
| + | |style="text-align:right;"|וכמו שיכפלו כ' די' בו' שנים וח' חדשים כן בזה הזמן יכפלו הצ"ה ליט‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Therefore it is said: divide 20 years into 3 parts. | ||
| + | |style="text-align:right;"|ולכן אמר חלק כ' שנים בג' חלקים | ||
|- | |- | ||
| | | | ||
| Line 4,474: | Line 5,744: | ||
|- | |- | ||
| | | | ||
| − | ::100 | + | ::Divide 100 years into as many parts as the [number of] liṭra that one kikkar yields a year. |
::<math>\scriptstyle{\color{blue}{X=\frac{100}{a}}}</math> | ::<math>\scriptstyle{\color{blue}{X=\frac{100}{a}}}</math> | ||
|style="text-align:right;"|חלק ק' שנים בכל כך חלקים כמו הליט' שירויח הככר לשנה | |style="text-align:right;"|חלק ק' שנים בכל כך חלקים כמו הליט' שירויח הככר לשנה | ||
|- | |- | ||
| | | | ||
| − | :*{{#annot:years, for some liṭra|660|zBWq}} | + | :*{{#annot:years, for some liṭra|660|zBWq}}Example: one kikkar yields 8 liṭra a year. |
::In how many [years] will 45 liṭra be doubled? | ::In how many [years] will 45 liṭra be doubled? | ||
::<math>\scriptstyle\frac{8}{1\sdot100}=\frac{45}{X\sdot45}</math> | ::<math>\scriptstyle\frac{8}{1\sdot100}=\frac{45}{X\sdot45}</math> | ||
| Line 4,486: | Line 5,756: | ||
|- | |- | ||
| | | | ||
| + | ::Divide 100 years into 8 parts; the result is 12 years and 6 months. | ||
| + | |style="text-align:right;"|חלק ק' שנים בח' חלקים יבואו י"ב שנים וו' חדשים | ||
| + | |- | ||
| + | | | ||
| + | ::We find that the 45 liṭra are doubled in 12 years and 6 months. | ||
::<math>\scriptstyle{\color{blue}{X=\frac{100}{8}=12+\frac{6}{12}}}</math> | ::<math>\scriptstyle{\color{blue}{X=\frac{100}{8}=12+\frac{6}{12}}}</math> | ||
| − | + | |style="text-align:right;"|נמצא שבי"ב שנים וו' חדשים יכפלו מ"ה ליט‫' | |
| − | |style="text-align:right;"| | ||
| − | נמצא שבי"ב שנים וו' חדשים יכפלו מ"ה ליט‫' | ||
|- | |- | ||
| | | | ||
| − | ::Check: | + | ::<span style=color:green>'''Check:'''</span> This calculation is as follows: it is known that the kikkar is doubled at the same time that any part of the kikkar is doubled, whether it is 10 or any number. |
| − | |style="text-align:right;"|וזה החשבון יוצא כך ידוע כי בזמן שיכפול הככר באותו הזמן עצמו יכפול כל חלק וחלק מן הככר בין לחשבון י' בין לכל חשבון שיהיה | + | |style="text-align:right;"|וזה החשבון יוצא כך ידוע כי בזמן שיכפול הככר באותו הזמן עצמו יכפול כל חלק וחלק מן הככר בין לחשבון י' בין לכל חשבון שיהיה |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | + | ::To make the calculation easier, consider the 45 liṭra as if they are a whole kikkar. | |
| − | + | |style="text-align:right;"|וכדי להקל החשבון חשוב המ"ה ליט' יהיו ככר שלם | |
| − | |||
| − | |style="text-align:right;"| | ||
| − | |||
|- | |- | ||
| | | | ||
| − | :: | + | ::At 8 liṭra for one kikkar a year, the kikkar is doubled in 12 and a half years. |
| − | + | |style="text-align:right;"|ולחשבון ח' ליט' הככר לשנה יכפול הככר בי"ב שנים וחצי | |
| − | |style="text-align:right;"| | ||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| + | ::Because 12 and a half times 8 liṭra is 100 liṭra. | ||
| + | ::<math>\scriptstyle{\color{blue}{8\sdot\left(12+\frac{6}{12}\right)=8\sdot\left(12+\frac{1}{2}\right)=100}}</math> | ||
| + | |style="text-align:right;"|כי י"ב פעמים וחצי ח' ליט' הם ק' ליט‫' | ||
| + | |- | ||
| + | | | ||
| + | ::So, as the kikkar is doubled in 12 and a half years, the 45 liṭra are doubled in 12 and a half years. | ||
| + | |style="text-align:right;"|אם כן כמו שהככר יכפל בי"ב שנים וחצי כן המ"ה ליט' יכפלו בי"ב שנים וחצי | ||
| + | |- | ||
| + | | | ||
| + | ::Therefore, say: divide 100 into 8 parts; the result is 12 and a half years. | ||
| + | |style="text-align:right;"|ולכן אמ' אמור חלק ק' בח' חלקים יבוא י"ב שנים וחצי | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:liṭra for some months|666|jgz4}}If one says: one kikkar yields 29 liṭra, 13 dinar, and 6 pešuṭim a year. | ||
| + | :How many liṭra will yield the same in 7 months? | ||
| + | :<math>\scriptstyle\frac{29+\frac{13}{20}+\frac{6}{20\sdot12}}{12\sdot100}=\frac{29+\frac{13}{20}+\frac{6}{20\sdot12}}{7\sdot X}</math> | ||
| + | |style="text-align:right;"|אם יאמר אדם הככר ירויח בשנה כ"ט ליט' וי"ג די' ו' פשו‫'<br> | ||
| + | כמה ליט' ירויחו כהם בז' חדשים{{#annotend:jgz4}} | ||
| + | |- | ||
| + | | | ||
| + | ::Multiply the months of the year by the number of liṭra, i.e. by 100 liṭra, and divide them into as many parts as the [number of the] months we wish to know, i.e. 7 months. | ||
| + | |style="text-align:right;"|תרבה חדשי השנה עם הסך הליט' דהינו עם ק' ליט' וחלקם בכל כך חלקים כמו החדשים שנרצה לידע דהיינו הז' חדשים | ||
| + | |- | ||
| + | | | ||
| + | ::Say: 12 times 100; the result is one thousand and 200. | ||
| + | |style="text-align:right;"|ואמור י"ב פעמי' ק' יבואו אלף ור‫' | ||
| + | |- | ||
| + | | | ||
| + | ::Divide it by 7; the result is 171 liṭra, 8 dinar, 6 pešuṭim and 6 parts of 7 of one pašuṭ. | ||
| + | ::<math>\scriptstyle{\color{blue}{X=\frac{12\sdot100}{7}=\frac{1200}{7}=171+\frac{8}{20}+\frac{6+\frac{6}{7}}{20\sdot12}}}</math> | ||
| + | |style="text-align:right;"|וחלקם על ז' יבואו קע"א ליט' וח' די' ו' פשו' וו' חלקים מז' בפשו‫' | ||
| + | |- | ||
| + | | | ||
| + | ::So, they yield in 7 months as much as the kikkar yields in a year. | ||
| + | |style="text-align:right;"|הרי שירויחו בז' חדשים כמו שירויח [הככר] א' שנה | ||
| + | |- | ||
| + | |Know that this calculation is a general calculation, so you can say that the kikkar yields 20, or 30, or 25 liṭra a year and so on for any number you want. | ||
|style="text-align:right;"|ודע כי זה הוא חשבון חשבון כללי וכן תוכל לאמר הככר ירויח לשנה כ' ליט' או ל' או כ"ה וכן בכל עניין שתרצה הכל ‫[...] | |style="text-align:right;"|ודע כי זה הוא חשבון חשבון כללי וכן תוכל לאמר הככר ירויח לשנה כ' ליט' או ל' או כ"ה וכן בכל עניין שתרצה הכל ‫[...] | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:years, for some dinar|660|DVte}}one liṭra | + | *{{#annot:years, for some dinar|660|DVte}}if one says: I lent one liṭra at 2 and a half pešuṭim a month. |
| − | :In how many [years] will | + | :In how many [years] it will be doubled? |
:<math>\scriptstyle\frac{2+\frac{1}{2}}{1\sdot20}=\frac{12\sdot a}{12\sdot X\sdot a}</math> | :<math>\scriptstyle\frac{2+\frac{1}{2}}{1\sdot20}=\frac{12\sdot a}{12\sdot X\sdot a}</math> | ||
|style="text-align:right;"|אם יאמר אדם הלויתי הליט' לחשבון ב' פשו' וחצי לחדש<br> | |style="text-align:right;"|אם יאמר אדם הלויתי הליט' לחשבון ב' פשו' וחצי לחדש<br> | ||
| Line 4,527: | Line 5,824: | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{X=\frac{20}{2+\frac{1}{2}}}}</math> | + | :Divide 20 years into as many parts as the [number of pešuṭim] that the liṭra yields a month. |
| + | :<math>\scriptstyle{\color{blue}{X=\frac{20}{2+\frac{1}{2}}}}</math> | ||
|style="text-align:right;"|חלק כ' שנים בכל כך חלקים כמו המעו' שירויח הליט' לחדש | |style="text-align:right;"|חלק כ' שנים בכל כך חלקים כמו המעו' שירויח הליט' לחדש | ||
|- | |- | ||
| | | | ||
| − | :*{{#annot:years, for some dinar|660|ITxm}} | + | :*{{#annot:years, for some dinar|660|ITxm}}Example: I lent 20 dinar at 2 and a half pešuṭim for one liṭra a month. |
| − | :: | + | ::You wish to know: in how many [years] will they be doubled? |
::<math>\scriptstyle\frac{2+\frac{1}{2}}{1\sdot20}=\frac{12\sdot20}{12\sdot X\sdot20}</math> | ::<math>\scriptstyle\frac{2+\frac{1}{2}}{1\sdot20}=\frac{12\sdot20}{12\sdot X\sdot20}</math> | ||
|style="text-align:right;"|המשל בזה הלוויתי כ' דינ' לחשבון ב' פשו' וחצי הליט' לחדש ותרצה לידע בכמה זמן יכפלו{{#annotend:ITxm}} | |style="text-align:right;"|המשל בזה הלוויתי כ' דינ' לחשבון ב' פשו' וחצי הליט' לחדש ותרצה לידע בכמה זמן יכפלו{{#annotend:ITxm}} | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{X=\frac{20}{2+\frac{1}{2}}=8}}</math> | + | ::Divide 20 dinar by 2 and a half; the result is 8 years. |
| − | |style="text-align:right;"|חלק כ' דינ' בב' וחצי יבואו ח' שנים | + | ::<math>\scriptstyle{\color{blue}{X=\frac{20}{2+\frac{1}{2}}=8}}</math> |
| − | + | |style="text-align:right;"|חלק כ' דינ' בב' וחצי יבואו ח' שנים | |
|- | |- | ||
| | | | ||
| − | ::Check: | + | ::We find that they are doubled in 8 years. |
| + | |style="text-align:right;"|נמצא שבח' שנים יכפלו זה | ||
| + | |- | ||
| + | | | ||
| + | ::<span style=color:green>'''Check:'''</span> This calculation is as follows: it is already known that at 2 and a half pešuṭim for one liṭra a month, the result is 2 and a half dinar a year. | ||
|style="text-align:right;"|וזה החשבון יוצא כך כבר ידוע כי לחשבון ב' פשו' וחצי הליט' יבא בשנה ב' דינ' וחצי | |style="text-align:right;"|וזה החשבון יוצא כך כבר ידוע כי לחשבון ב' פשו' וחצי הליט' יבא בשנה ב' דינ' וחצי | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> So say: if 2 and a half dinar yield 20 dinar a year, in how many [years] will 20 dinar be doubled? | |
| + | :<math>\scriptstyle{\color{blue}{\left(2+\frac{1}{2}\right):1=20:X}}</math> | ||
|style="text-align:right;"|אמור אם כן אם ב' דינ' וחצי יהיו בא' שנה כ' דינ' כ' דינ' דהיינו שיכפלו בכמה זמן יהיו | |style="text-align:right;"|אמור אם כן אם ב' דינ' וחצי יהיו בא' שנה כ' דינ' כ' דינ' דהיינו שיכפלו בכמה זמן יהיו | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{X=\frac{1\sdot20}{2+\frac{1}{2}}=\frac{20}{2+\frac{1}{2}}=8}}</math> | + | ::Say: 20 times 1 is 20. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אמור כ' פעמים א' כ‫' |
| − | חלקם בב' וחצי יבואו ח‫' | + | |- |
| − | הרי שבח' שנים יכפול | + | | |
| + | ::Divide it by 2 and a half; the result is 8 | ||
| + | ::<math>\scriptstyle{\color{blue}{X=\frac{1\sdot20}{2+\frac{1}{2}}=\frac{20}{2+\frac{1}{2}}=8}}</math> | ||
| + | |style="text-align:right;"|חלקם בב' וחצי יבואו ח‫' | ||
| + | |- | ||
| + | | | ||
| + | ::So, they are doubled in 8 years. | ||
| + | |style="text-align:right;"|הרי שבח' שנים יכפול | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:for some liṭra a day|638|kEfW}} | + | *{{#annot:for some liṭra a day|638|kEfW}}If a man says: I lent one liṭra at so and so a month. |
:How much will [100] liṭra yield a day? | :How much will [100] liṭra yield a day? | ||
:<math>\scriptstyle\frac{a}{30\sdot1}=\frac{X}{1\sdot100}</math> | :<math>\scriptstyle\frac{a}{30\sdot1}=\frac{X}{1\sdot100}</math> | ||
| Line 4,563: | Line 5,873: | ||
|- | |- | ||
| | | | ||
| − | : | + | :Multiply 3 and a half by the money of the interest. |
| − | + | :<math>\scriptstyle{\color{blue}{X=\left(3+\frac{1}{3}\right)\sdot a}}</math> | |
|style="text-align:right;"|תרבה ג' ושליש כנגד מעו' הרבית | |style="text-align:right;"|תרבה ג' ושליש כנגד מעו' הרבית | ||
|- | |- | ||
| | | | ||
| − | : | + | :{{#annot:for some liṭra a day|638|FCzK}}Example: I lent one liṭra at 3 pešuṭim a month. |
| − | : | + | :You want to know how much will 100 liṭra yield a day? |
| − | + | :<math>\scriptstyle\frac{3}{30\sdot1}=\frac{X}{1\sdot100}</math> | |
|style="text-align:right;"|המשל בזה הלוותי הליט' לחשבון ג' פשו' הליט' לחדש<br> | |style="text-align:right;"|המשל בזה הלוותי הליט' לחשבון ג' פשו' הליט' לחדש<br> | ||
ותרצה לדעת כמה ירויחו היום ק' ליט‫'{{#annotend:FCzK}} | ותרצה לדעת כמה ירויחו היום ק' ליט‫'{{#annotend:FCzK}} | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{X=\left(3+\frac{1}{3}\right)\sdot3=10}}</math> | + | :Multiply 3 and a third by 3 and say: 3 and a third times 3 is 10 pešuṭim. We find that 100 liṭra yield 10 pešuṭim a day. |
| + | :<math>\scriptstyle{\color{blue}{X=\left(3+\frac{1}{3}\right)\sdot3=10}}</math> | ||
|style="text-align:right;"|תרבה ג' ושליש עם ג' ואמור ג' ושליש פעמי' ג' הם י' פשו‫'<br> | |style="text-align:right;"|תרבה ג' ושליש עם ג' ואמור ג' ושליש פעמי' ג' הם י' פשו‫'<br> | ||
נמצא שק' ליט' ירויחו ביום אחד י' פשו‫' | נמצא שק' ליט' ירויחו ביום אחד י' פשו‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | :You can know this because one kikkar yields 15 liṭra a year at 3 pešuṭim a month for one liṭra. |
|style="text-align:right;"|וזה החשבון תוכל לדעת בעניין זה כי לחשבון ג' פשו' הליט' לחדש יבא הככר לשנה ט"ו ליט‫' | |style="text-align:right;"|וזה החשבון תוכל לדעת בעניין זה כי לחשבון ג' פשו' הליט' לחדש יבא הככר לשנה ט"ו ליט‫' | ||
|- | |- | ||
| | | | ||
| − | ::::<math>\scriptstyle{\color{blue}{\frac{2\sdot15}{3}=\frac{30}{3}=10}}</math> | + | :From the year you can deduce how much will be earned a day. Double it and take a third. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ומהשנה תוכל לדעת כמה יבא ביום ותכפול אותו ותפוש השליש |
| − | ואם תכפול ט"ו יהיו ל' ותפוש השליש והם י‫'<br> | + | |- |
| − | או תפוש הרוב מט"ו והם י‫' | + | | |
| − | וכן יבא הככר ליום כשתלווה ליט' לחשבון ג' פשו' הליט' בחדש וכן לעולם | + | :If you double 15, it is 30. Take a third; it is 10 [pešuṭim a day]. |
| + | :<math>\scriptstyle{\color{blue}{\frac{2\sdot15}{3}=\frac{30}{3}=10}}</math> | ||
| + | |style="text-align:right;"|ואם תכפול ט"ו יהיו ל' ותפוש השליש והם י‫'<br> | ||
| + | או תפוש הרוב מט"ו והם י‫' | ||
| + | |- | ||
| + | | | ||
| + | :This is what one kikkar yields a day, when you lent one liṭra at 3 pešuṭim a month | ||
| + | |style="text-align:right;"|וכן יבא הככר ליום כשתלווה ליט' לחשבון ג' פשו' הליט' בחדש | ||
| + | |- | ||
| + | | | ||
| + | :And so on. | ||
| + | |style="text-align:right;"|וכן לעולם | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:for one liṭra a month|638|cqZ2}} | + | *{{#annot:for one liṭra a month|638|cqZ2}}If a man say: so and so liṭra yield one pašuṭ a day. |
| − | :How | + | :How much will one liṭra yield a month? |
:<math>\scriptstyle\frac{1}{1\sdot a}=\frac{X}{30\sdot1}</math> | :<math>\scriptstyle\frac{1}{1\sdot a}=\frac{X}{30\sdot1}</math> | ||
|style="text-align:right;"|אם יאמר אדם כל כך ליט' ירויחו א' פשו' ביום<br> | |style="text-align:right;"|אם יאמר אדם כל כך ליט' ירויחו א' פשו' ביום<br> | ||
| Line 4,598: | Line 5,920: | ||
|- | |- | ||
| | | | ||
| − | : | + | :Divide 30 pešuṭim by as many liṭra that yield one pašuṭ a day. |
| − | + | :<math>\scriptstyle{\color{blue}{X=\frac{30}{a}}}</math> | |
|style="text-align:right;"|חלק ל' פשו' בכל כך חלקים כמו הליט' שירוויחו ביום א' פשו‫' | |style="text-align:right;"|חלק ל' פשו' בכל כך חלקים כמו הליט' שירוויחו ביום א' פשו‫' | ||
|- | |- | ||
| | | | ||
| − | + | *{{#annot:for one liṭra a month|638|QVqt}}Example: 12 liṭra yield one pašuṭ a day. | |
| − | : | + | :You wish to know how much will one liṭra yield a month. |
| − | + | :<math>\scriptstyle\frac{1}{1\sdot12}=\frac{X}{30\sdot1}</math> | |
|style="text-align:right;"|המשל בזה י"ב ליט' ירויחו ליום א' פשו‫'<br> | |style="text-align:right;"|המשל בזה י"ב ליט' ירויחו ליום א' פשו‫'<br> | ||
ותרצה לידע כמה יגיע לליט' בחדש{{#annotend:QVqt}} | ותרצה לידע כמה יגיע לליט' בחדש{{#annotend:QVqt}} | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{X=\frac{30}{12}=2+\frac{1}{2}}}</math> | + | :Divide 30 pešuṭim into 12 parts; the result is 2 and a half. We find that the liṭra yields 2 pešuṭim and a half. |
| + | :<math>\scriptstyle{\color{blue}{X=\frac{30}{12}=2+\frac{1}{2}}}</math> | ||
|style="text-align:right;"|חלק ל' פשו' לי"ב חלקים יבואו ב' וחצי<br> | |style="text-align:right;"|חלק ל' פשו' לי"ב חלקים יבואו ב' וחצי<br> | ||
נמצא שיבא לחשבון ב' פשו' וחצי הליט‫' | נמצא שיבא לחשבון ב' פשו' וחצי הליט‫' | ||
|- | |- | ||
| | | | ||
| + | :This is the result, since 12 liṭra yield each day one pašuṭ, so they yield in 30 days, which is a month, [30] pešuṭim. | ||
|style="text-align:right;"|וזה החשבון יוצא כך שאחרי שהי"ב ליט' מרויחים בכל יום א' פשו‫'<br> | |style="text-align:right;"|וזה החשבון יוצא כך שאחרי שהי"ב ליט' מרויחים בכל יום א' פשו‫'<br> | ||
אם כן מרויחים בל' יום שהוא א' חדש א' פשו‫' | אם כן מרויחים בל' יום שהוא א' חדש א' פשו‫' | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | === Find a Number Problems === | + | === <span style=color:green>Find a Number Problems</span> === |
| | | | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:repeated subtraction|618|QPoG}} | + | *{{#annot:repeated subtraction|618|QPoG}}If one asks you: a number, you subtract a tenth from it, then you subtract again a tenth from the remainder, and 100 are left. |
:How much was the original number before subtracting the two tenths?<br> | :How much was the original number before subtracting the two tenths?<br> | ||
:<math>\scriptstyle X-\frac{1}{10}X-\left[\frac{1}{10}\sdot\left(X-\frac{1}{10}X\right)\right]=100</math> | :<math>\scriptstyle X-\frac{1}{10}X-\left[\frac{1}{10}\sdot\left(X-\frac{1}{10}X\right)\right]=100</math> | ||
| − | |style="text-align:right;"|אם ישאלך אדם חשבון שהסרות ממנו הי' ומן הנשאר הסרות ממנו העשירית פעם שנית ונשארו ק‫'<br> | + | |style="text-align:right;"|<big>אם ישאלך אדם חשבון שהסרות ממנו הי'</big> ומן הנשאר הסרות ממנו העשירית פעם שנית ונשארו ק‫'<br> |
כמה היה כל החשבון בטרם שהסרת ממנו ב' העשיריות{{#annotend:QPoG}} | כמה היה כל החשבון בטרם שהסרת ממנו ב' העשיריות{{#annotend:QPoG}} | ||
|- | |- | ||
| | | | ||
| − | :: | + | :Do this way: |
| − | + | |style="text-align:right;"|עשה על זה הדרך | |
| − | |style="text-align:right;"|עשה על זה הדרך | ||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''False position:'''</span> Say: if the number is 100 and you subtract the 2-tenths from it, 81 remains. | |
| − | + | :<math>\scriptstyle{\color{blue}{X=100}}</math> | |
| − | |style="text-align:right;"|אמור | + | |style="text-align:right;"|אמור אלו על זה הדרך<br> |
| + | אלו היה החשבון ק' ופחתת ממנו ב' עשיריות ישארו פ"א | ||
|- | |- | ||
| | | | ||
| − | : | + | :The tenth of 100 is 10; ninety remains. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כיצד העשירית מק' והם י' נשארו תשעים |
| − | |||
|- | |- | ||
| | | | ||
| − | :: | + | :You subtract again the tenth from 90; 81 remains. |
| + | |style="text-align:right;"|חסרת עוד העשירית מצ' והם נשארו פ"א | ||
| + | |- | ||
| | | | ||
| + | :So, when 81 remains after you subtract 2-tenths, there was 100 at the beginning. | ||
| + | |style="text-align:right;"|הרי שבעת שנשארו פ"א אחרי הסרת ב' עשיריות היו ק' בראשונה | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | :<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle100-\left(\frac{1}{10}\sdot100\right)-\left[\frac{1}{10}\sdot\left[100-\left(\frac{1}{10}\sdot100\right)\right]\right]&\scriptstyle=100-10-\left[\frac{1}{10}\sdot\left(100-10\right)\right]\\&\scriptstyle=90-\left(\frac{1}{10}\sdot90\right)=81\\\end{align}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :<span style=color:green>'''Rule of Three:'''</span> Say: if 81 remains from [100] after you subtract 2-tenths from it, 2-tenths of what is 19? | ||
| + | :<math>\scriptstyle{\color{blue}{100-81=19}}</math> | ||
| + | :<math>\scriptstyle{\color{blue}{81:100=19:a}}</math> | ||
| + | |style="text-align:right;"|אמור [אם כן אם בחשבו' שנשארו פ"א אחר שהסרת ממנו הב' עשיריו' כמה היו הב' עשרייו' י"ט]‫<ref>MS Ithaca om.</ref> | ||
| + | |- | ||
| + | | | ||
| + | :100 times 19 is one thousand and 900. | ||
| + | |style="text-align:right;"|אם כן ק' פעמים <s>ה</s> י"ט הם אלף ותת"ק | ||
|- | |- | ||
| | | | ||
| + | :Divide by 81; it is 23 and 37 parts of 81. | ||
| + | :<math>\scriptstyle{\color{blue}{a=\frac{100\sdot19}{81}=\frac{1900}{81}=23+\frac{37}{81}}}</math> | ||
| + | |style="text-align:right;"|חלק בפ"א יהיו כ"ג ול"ז חלקים מפ"א | ||
| + | |- | ||
| + | | | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{X=123+\frac{37}{81}}}</math> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :Subtract the tenths from it, which are 12 and 28 parts of 81: | ||
|style="text-align:right;"|הסר מהם העשיריות שהם י"ב וכ"ח חלקים מפ"א | |style="text-align:right;"|הסר מהם העשיריות שהם י"ב וכ"ח חלקים מפ"א | ||
|- | |- | ||
| | | | ||
| − | :::<math>\scriptstyle{\color{blue}{\frac{1}{10}\sdot120=12}}</math> | + | ::The tenth of 120 is 12. |
| + | ::<math>\scriptstyle{\color{blue}{\frac{1}{10}\sdot120=12}}</math> | ||
|style="text-align:right;"|כיצד העשירית מק"כ הם י"ב | |style="text-align:right;"|כיצד העשירית מק"כ הם י"ב | ||
|- | |- | ||
| | | | ||
| − | :::<math>\scriptstyle{\color{blue}{\frac{1}{10}\sdot\left(3+\frac{37}{81}\right)=\frac{1}{10}\sdot\frac{280}{81}=\frac{28}{81}}}</math> | + | ::3 and 37 parts of 81 are 280 parts of 81; their tenth is 28 parts of 81. |
| + | ::<math>\scriptstyle{\color{blue}{\frac{1}{10}\sdot\left(3+\frac{37}{81}\right)=\frac{1}{10}\sdot\frac{280}{81}=\frac{28}{81}}}</math> | ||
|style="text-align:right;"|והג' והל"ז חלקים מפ"א הם ר"פ חלקים מפ"א<br> | |style="text-align:right;"|והג' והל"ז חלקים מפ"א הם ר"פ חלקים מפ"א<br> | ||
והעשירית מהם הם כ"ח חלקים מפ"א | והעשירית מהם הם כ"ח חלקים מפ"א | ||
|- | |- | ||
| | | | ||
| − | : | + | :So, the first tenth is 12 and 28 parts of 81. |
| + | :<math>\scriptstyle{\color{blue}{12+\frac{28}{81}}}</math> | ||
|style="text-align:right;"|הרי שהמעשר הראשון היה י"ב וכ"ח חלקים מפ"א | |style="text-align:right;"|הרי שהמעשר הראשון היה י"ב וכ"ח חלקים מפ"א | ||
|- | |- | ||
| | | | ||
| − | : | + | :108 and 252 parts of 81 remain, which is 111 and 9 parts of 81. |
| − | |||
|style="text-align:right;"|נשארו ק"ח ורנ"ב חלקי' מפ"א<br> | |style="text-align:right;"|נשארו ק"ח ורנ"ב חלקי' מפ"א<br> | ||
שהם קי"א וט' חלקי' מפ"א | שהם קי"א וט' חלקי' מפ"א | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | :<math>\scriptstyle{\color{blue}{\left(123+\frac{37}{81}\right)-\left[\frac{1}{10}\sdot\left(123+\frac{37}{81}\right)\right]=\left(123+\frac{37}{81}\right)-\left(12+\frac{28}{81}\right)=108+\frac{252}{81}=111+\frac{9}{81}}}</math> | ||
|- | |- | ||
| | | | ||
| − | : | + | :Subtract from it another tenth, which is 11 and 9 parts of 81; 100 remains: |
|style="text-align:right;"|הסר מהם עוד העשירית שהם י"א וט' חלקים מפ"א<br> | |style="text-align:right;"|הסר מהם עוד העשירית שהם י"א וט' חלקים מפ"א<br> | ||
ישארו ק‫' | ישארו ק‫' | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | :<math>\scriptstyle{\color{blue}{\begin{align}&\scriptstyle\left(123+\frac{37}{81}\right)-\left[\frac{1}{10}\sdot\left(123+\frac{37}{81}\right)\right]-\left[\frac{1}{10}\sdot\left[\left(123+\frac{37}{81}\right)-\left[\frac{1}{10}\sdot\left(123+\frac{37}{81}\right)\right]\right]\right]\\&\scriptstyle=111+\frac{9}{81}-\left[\frac{1}{10}\sdot\left(111+\frac{9}{81}\right)\right]\\&\scriptstyle=111+\frac{9}{81}-\left(11+\frac{9}{81}\right)=100\\\end{align}}}</math> | ||
|- | |- | ||
| | | | ||
| − | :::<math>\scriptstyle{\color{blue}{\frac{1}{10}\sdot110=11}}</math> | + | ::The tenth of 110 is 11. |
| + | ::<math>\scriptstyle{\color{blue}{\frac{1}{10}\sdot110=11}}</math> | ||
|style="text-align:right;"|כיצד העשיריות מק"י הוא י"א | |style="text-align:right;"|כיצד העשיריות מק"י הוא י"א | ||
|- | |- | ||
| | | | ||
| − | :::<math>\scriptstyle{\color{blue}{\frac{1}{10}\sdot\left(1+\frac{9}{81}\right)=\frac{1}{10}\sdot\frac{90}{81}=\frac{9}{81}}}</math> | + | ::[1] and 9 parts of 81 are 90 parts of 81; their tenth is 9 parts of 81. |
| − | |style="text-align:right;"| | + | ::<math>\scriptstyle{\color{blue}{\frac{1}{10}\sdot\left(1+\frac{9}{81}\right)=\frac{1}{10}\sdot\frac{90}{81}=\frac{9}{81}}}</math> |
| + | |style="text-align:right;"|וה[א'] והט' חלקי' מפ"א הם צ' חלקי' מפ"א<br> | ||
והעשירית מהם הוא ט' חלקי' מפ"א | והעשירית מהם הוא ט' חלקי' מפ"א | ||
|- | |- | ||
| | | | ||
| − | :: | + | :The first tenth is 12 and 28 parts of 81. |
| − | |style="text-align:right;"|הרי שהמעשר הראשון היה י"ב וכ"ח חלקים מפ"א | + | :<math>\scriptstyle{\color{blue}{12+\frac{28}{81}}}</math> |
| + | |style="text-align:right;"|הרי ‫<ref>42v</ref>שהמעשר הראשון היה י"ב וכ"ח חלקים מפ"א | ||
|- | |- | ||
| | | | ||
| − | :: | + | :111 and 9 parts of 81 remain. |
| + | :<math>\scriptstyle{\color{blue}{111+\frac{9}{81}}}</math> | ||
|style="text-align:right;"|ונשארו קי"א וט' חלקי' מפ"א | |style="text-align:right;"|ונשארו קי"א וט' חלקי' מפ"א | ||
|- | |- | ||
| | | | ||
| − | :: | + | :The second tenth is 11 and 9 parts of 81. |
| + | :<math>\scriptstyle{\color{blue}{11+\frac{9}{81}}}</math> | ||
|style="text-align:right;"|והמעשר השני היה י"א וט' חלקי' מפ"א | |style="text-align:right;"|והמעשר השני היה י"א וט' חלקי' מפ"א | ||
|- | |- | ||
| | | | ||
| − | : | + | :100 remains. |
|style="text-align:right;"|ונשארו ק‫' | |style="text-align:right;"|ונשארו ק‫' | ||
|- | |- | ||
| | | | ||
| − | *{{#annot: | + | *{{#annot:(3+⅓)×(4+¼)|17|PNQv}}How much are 3 and a third times 4 and a quarter? |
:<math>\scriptstyle\left(3+\frac{1}{3}\right)\times\left(4+\frac{1}{4}\right)</math> | :<math>\scriptstyle\left(3+\frac{1}{3}\right)\times\left(4+\frac{1}{4}\right)</math> | ||
| − | |style="text-align:right;"|אם תרצה לדעת ג' ושליש פעמים ד' ורביע כמה עולי‫'{{#annotend:PNQv}} | + | |style="text-align:right;"|<big>אם תרצה לדעת ג' ושליש פעמים ד'</big> ורביע כמה עולי‫'{{#annotend:PNQv}} |
| + | |- | ||
| + | | | ||
| + | :Convert the 3 and a third into thirds: | ||
| + | |style="text-align:right;"|עשה מן הג' ושליש כלם שלישיות | ||
| + | |- | ||
| + | | | ||
| + | :Say: 3 times 3 is 9; plus a third, it is 10. Write it down. | ||
| + | :<math>\scriptstyle{\color{blue}{3+\frac{1}{3}=\frac{\left(3\sdot3\right)+1}{3}=\frac{10}{3}}}</math> | ||
| + | |style="text-align:right;"|ואמור ג' פעמים ג' ט' וא' שליש הרי י' ותכתבם | ||
| + | |- | ||
| + | | | ||
| + | :Convert the 4 and a quarter into quarters: | ||
| + | |style="text-align:right;"|אחרי כן עשה מן הד' ורביע כלם רביעיות | ||
|- | |- | ||
| | | | ||
| − | :: | + | :Say: 4 times 4 is 16; plus a quarter, it is 17. Write it down. |
| − | + | :<math>\scriptstyle{\color{blue}{4+\frac{1}{4}=\frac{\left(4\sdot4\right)+1}{4}=\frac{17}{4}}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואמור ד' פעמים ד' י"ו וא' רביע הרי י"ז ותכתבם |
| − | ואמור | ||
|- | |- | ||
| | | | ||
| − | : | + | :So, we have 10 thirds and 17 quarters. |
| − | + | |style="text-align:right;"|הרי שיש לנו י' שלישיות וי"ז רביעיות | |
| − | |style="text-align:right;"| | ||
| − | |||
|- | |- | ||
| | | | ||
| − | :: | + | :Multiply them by each other and say: 10 times 17 is 170. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|תרבה אותם זה עם זה ואמור י' פעמי' י"ז הם ק"ע |
| − | תרבה אותם זה עם זה ואמור י' פעמי' י"ז הם ק"ע | ||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| + | :Divide it by 3 times 4, which is 12; it is 14 and 2 parts of 12, which is a sixth. | ||
| + | |style="text-align:right;"|וחלקם בג' פעמים ד' שהם י"ב הרי י"ד וב' חלקי' מי"ב שהם א' שתות | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | :<math>\scriptstyle{\color{blue}{\left(3+\frac{1}{3}\right)\times\left(4+\frac{1}{4}\right)=\frac{10}{3}\sdot\frac{17}{4}=\frac{10\sdot17}{3\sdot4}=\frac{170}{12}=14+\frac{2}{12}=14+\frac{1}{6}}}</math> | ||
| + | |- | ||
| + | |Do this way for all. | ||
|style="text-align:right;"|ועל זה הדרך תעשה כלם | |style="text-align:right;"|ועל זה הדרך תעשה כלם | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | === Find the Price - Cubits of Cloth === | + | === <span style=color:green>Find the Price - Cubits of Cloth</span> === |
| | | | ||
|- | |- | ||
| | | | ||
| − | *{{#annot: | + | *{{#annot:cloth|629|eLS7}}If you wish to know how much are 3 cubits of cloth plus one third, a quarter, and a fifth for 4 pešuṭim plus a quarter, a fifth, and a sixth? |
:<math>\scriptstyle\left(3+\frac{1}{3}+\frac{1}{4}+\frac{1}{5}\right)\times\left(4+\frac{1}{4}+\frac{1}{5}+\frac{1}{6}\right)</math> | :<math>\scriptstyle\left(3+\frac{1}{3}+\frac{1}{4}+\frac{1}{5}\right)\times\left(4+\frac{1}{4}+\frac{1}{5}+\frac{1}{6}\right)</math> | ||
| − | |style="text-align:right;"|ואם תרצה לדעת ג' אמות בגד ושליש ורביע וחומש לחשבון ד"פ ורביע וחומש ושתות כמה יבואו{{#annotend:eLS7}} | + | |style="text-align:right;"|<big>ואם תרצה לדעת ג' אמות בגד ושליש ורביע</big> וחומש לחשבון ד"פ ורביע וחומש ושתות כמה יבואו{{#annotend:eLS7}} |
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\left(\frac{1}{3}\sdot60\right)+\left(\frac{1}{4}\sdot60\right)+\left(\frac{1}{5}\sdot60\right)=20+15+12=47}}</math> | + | :Say: a third, a quarter and a fifth are found in 60. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אמור שליש ורביע וחומש ימצאו בס‫' |
| + | |- | ||
| + | | | ||
| + | :The third is 20; the quarter is 15; the fifth is 12; their total is 47. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(\frac{1}{3}\sdot60\right)+\left(\frac{1}{4}\sdot60\right)+\left(\frac{1}{5}\sdot60\right)=20+15+12=47}}</math> | ||
| + | |style="text-align:right;"|השליש כ' הרביע ט"ו החומש י"ב וכללם מ"ז | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{3+\frac{1}{3}+\frac{1}{4}+\frac{1}{5}=3+\frac{47}{60}}}</math> | + | :As if the question is: 3 cubits and 47 parts of 60. |
| + | :<math>\scriptstyle{\color{blue}{3+\frac{1}{3}+\frac{1}{4}+\frac{1}{5}=3+\frac{47}{60}}}</math> | ||
|style="text-align:right;"|וכאלו השאילה היא ג' אמות ומ"ז חלקים מס‫' | |style="text-align:right;"|וכאלו השאילה היא ג' אמות ומ"ז חלקים מס‫' | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\left(\frac{1}{4}\sdot60\right)+\left(\frac{1}{5}\sdot60\right)+\left(\frac{1}{6}\sdot60\right)=15+12+10=37}}</math> | + | :Also, a quarter, a fifth and a sixth are found in 60. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכן רביע וחומש ושתות ימצאו בס‫' |
| + | |- | ||
| + | | | ||
| + | :The quarter is 15; the fifth is 12; the sixth is 10; their total is 37. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(\frac{1}{4}\sdot60\right)+\left(\frac{1}{5}\sdot60\right)+\left(\frac{1}{6}\sdot60\right)=15+12+10=37}}</math> | ||
| + | |style="text-align:right;"|הרביע ט"ו והחומש י"ב והשתות י' וכללם ל"ז | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{4+\frac{1}{4}+\frac{1}{5}+\frac{1}{6}=4+\frac{37}{60}}}</math> | + | :As if it is said: at 4 pešuṭim and 37 parts of 60. |
| + | :<math>\scriptstyle{\color{blue}{4+\frac{1}{4}+\frac{1}{5}+\frac{1}{6}=4+\frac{37}{60}}}</math> | ||
|style="text-align:right;"|והוא כאלו אמר לחשבון ד"פ ול"ז חלקים מס‫' | |style="text-align:right;"|והוא כאלו אמר לחשבון ד"פ ול"ז חלקים מס‫' | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{3+\frac{1}{3}+\frac{1}{4}+\frac{1}{5}=3+\frac{47}{60}=\frac{\left(3\sdot60\right)+47}{60}=\frac{180+47}{60}=\frac{227}{60}}}</math> | + | :Write them as you see in the diagram. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ותכתבם כאשר תראם בצורה‫<ref>MS Ithaca marg.: חסר הצורה</ref> |
| + | |- | ||
| + | | | ||
| + | :Multiply the upper digits and say: 3 times 60 is 180. | ||
| + | |style="text-align:right;"|ותרבה האותיות העליונות ואמור ג' פעמי' ס' הם ק"פ | ||
| + | |- | ||
| + | | | ||
| + | :Add 47 to it; it is 227. Write it down. | ||
| + | |style="text-align:right;"|וצרף עמהם המ"ז הם רכ"ז ותכתבם | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | :<math>\scriptstyle{\color{blue}{3+\frac{1}{3}+\frac{1}{4}+\frac{1}{5}=3+\frac{47}{60}=\frac{\left(3\sdot60\right)+47}{60}=\frac{180+47}{60}=\frac{227}{60}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :Multiply the bottom digits and say: 4 times 60 is 240. | ||
| + | |style="text-align:right;"|ותרבה אחרי כן האותיות התחתונות ואמור ד' פעמי' ס' ר"מ | ||
| + | |- | ||
| + | | | ||
| + | :With the 37 written above, it is 277. Write it down. | ||
| + | |style="text-align:right;"|ול"ז הכתובים למעלה הרי רע"ז רכ"ז ותכתבם | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | :<math>\scriptstyle{\color{blue}{4+\frac{1}{4}+\frac{1}{5}+\frac{1}{6}=4+\frac{37}{60}=\frac{\left(4\sdot60\right)+37}{60}=\frac{240+37}{60}=\frac{277}{60}}}</math> | ||
|- | |- | ||
| | | | ||
| − | : | + | :Multiply 227 by 277; it is 62 thousand and 879. |
| − | |style="text-align:right;"|ותרבה | + | |style="text-align:right;"|ותרבה רכ"ז עם רע"ז יהיו ס"ב אלפי' ותתע"ט |
|- | |- | ||
| | | | ||
| − | :<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle\left(3+\frac{1}{3}+\frac{1}{4}+\frac{1}{5}\right)\times\left(4+\frac{1}{4}+\frac{1}{5}+\frac{1}{6}\right)&\scriptstyle=\frac{227}{60}\sdot\frac{277}{60} | + | :Divide it by 60 times 60, which is 3 thousand and 600; the result is 17 pešuṭim and one thousand and 879 parts of 3 thousand and 600 of one pašuṭ. |
| − | + | |style="text-align:right;"|חלקם בס' פעמי' ס' שהם ג' אלפים ות"ר יבאו י"ז פשוטי'‫<ref>י"ז פשוטי': MS Ithaca ה"פ</ref> ואלף ותרע"ט חלקים מג' אלפים ות"ר מן הפשוט | |
| − | + | |- | |
| + | | colspan="2"| | ||
| + | :<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle\left(3+\frac{1}{3}+\frac{1}{4}+\frac{1}{5}\right)\times\left(4+\frac{1}{4}+\frac{1}{5}+\frac{1}{6}\right)&\scriptstyle=\frac{227}{60}\sdot\frac{277}{60}=\frac{227\sdot277}{60\sdot60}\\&\scriptstyle=\frac{62879}{3600}=17+\frac{1679}{3600}\\\end{align}}}</math> | ||
|- | |- | ||
| | | | ||
| + | :Always do like this. | ||
|style="text-align:right;"|ועל זה הדרך תעשה לעולם | |style="text-align:right;"|ועל זה הדרך תעשה לעולם | ||
|- | |- | ||
| | | | ||
| − | === Interest and Discount Problem - Compound Interest === | + | === <span style=color:green>Interest and Discount Problem - Compound Interest</span> === |
| | | | ||
|- | |- | ||
| − | |{{#annot:WP|640|K4In}} | + | |{{#annot:WP|640|K4In}}Someone wants to sum several loans for different times. |
| − | |style="text-align:right;"|מי שרוצה לקבץ חשבונות הלואות רבות שנעשו בזמנים שונים{{#annotend:K4In}} | + | |style="text-align:right;"|<big>מי שרוצה לקבץ חשבונות הלואות</big> רבות שנעשו בזמנים שונים{{#annotend:K4In}} |
|- | |- | ||
| | | | ||
| − | : | + | :He sums the number of the months, then divides by the amount of money. |
|style="text-align:right;"|יקבץ מספר החדשים ויחלקם במספר המעות | |style="text-align:right;"|יקבץ מספר החדשים ויחלקם במספר המעות | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:WP|640|d6pd}}I lent 100 peraḥim for a month, 100 peraḥim for two months, 100 peraḥim for three months, 100 peraḥim for four months, and 100 peraḥim for five months | + | *{{#annot:WP|640|d6pd}}Example: I lent 100 peraḥim for a month, 100 peraḥim for two months, 100 peraḥim for three months, 100 peraḥim for four months, and 100 peraḥim for five months |
| − | |style="text-align:right;"|המשל בזה הרי שהלוית ק' פרחי' א' חדש וק' פרחי' הרי ב' חדשי' וק' פרחי' הרי ג' חדשי' וק' פרחי' הרי ד' חדשי' וק' פרחי' הרי ה' חדשי‫'{{#annotend:d6pd}} | + | |style="text-align:right;"|<big>המשל בזה</big> הרי שהלוית ק' פרחי' א' חדש וק' פרחי' הרי ב' חדשי' וק' פרחי' הרי ג' ‫<ref>43r</ref>חדשי' וק' פרחי' הרי ד' חדשי' וק' פרחי' הרי ה' חדשי‫'{{#annotend:d6pd}} |
| + | |- | ||
| + | | | ||
| + | :Do it this way: | ||
| + | |style="text-align:right;"|עשה על זה הדרך | ||
| + | |- | ||
| + | | | ||
| + | :Say: 1, 2, 3, 4, 5, which are the numbers of the months, are 15. | ||
| + | |style="text-align:right;"|אמור א' וב' וג' וד' וה' שהם חשבון החדשי' עולי' ט"ו | ||
|- | |- | ||
| | | | ||
| + | :Divide it by the number of the kikkar, which is 5; it is 3, so they are [5 kikkar] for 3 months. | ||
:<math>\scriptstyle{\color{blue}{\frac{1+2+3+4+5}{5}=\frac{15}{5}=3}}</math> | :<math>\scriptstyle{\color{blue}{\frac{1+2+3+4+5}{5}=\frac{15}{5}=3}}</math> | ||
| − | + | |style="text-align:right;"|חלק הסכום הככרים שהם ה' יהיו ג' וכאלו עמדו ג' חדשי‫' | |
| − | |style="text-align:right;"| | ||
| − | חלק הסכום הככרים שהם ה' יהיו ג' וכאלו עמדו ג' חדשי‫' | ||
|- | |- | ||
| − | | | + | |The same for any similar question. |
|style="text-align:right;"|וכן לכל חשבון כיוצא בזה | |style="text-align:right;"|וכן לכל חשבון כיוצא בזה | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | === Pricing Problems === | + | === <span style=color:green>Pricing Problems</span> === |
| | | | ||
| Line 4,818: | Line 6,228: | ||
*{{#annot:soil|629|PWsG}}One moiola's of soil are worth 12 staro. | *{{#annot:soil|629|PWsG}}One moiola's of soil are worth 12 staro. | ||
:If you buy one moiola's for one liṭra or more or less and you want to know how much one staro is worth | :If you buy one moiola's for one liṭra or more or less and you want to know how much one staro is worth | ||
| − | |style="text-align:right;"|המוייולו מן האדמה הם י"ב שטארו<br> | + | |style="text-align:right;"|‫<ref>This problem is missing in MS Ithaca</ref>המוייולו מן האדמה הם י"ב שטארו<br> |
אם תקנה המוייולו בעשרה ליט' או פחו' או יתר ותרצה לדעת כמה יבא השטארו{{#annotend:PWsG}} | אם תקנה המוייולו בעשרה ליט' או פחו' או יתר ותרצה לדעת כמה יבא השטארו{{#annotend:PWsG}} | ||
|- | |- | ||
| | | | ||
| − | : | + | :For any number you wish, take 20 [dinar] for each liṭra; and [12] pešuṭim for each dinar. |
| − | + | :<math>\scriptstyle{\color{OliveGreen}{X=12\sdot20\sdot a}}</math> | |
| − | + | |style="text-align:right;"|תפוש מכל ליט' כ' פשוטי' ומכל די' א' פשוט' וכן לכל חשבו' שתרצה | |
| − | |style="text-align:right;"|תפוש מכל ליט' כ | ||
|- | |- | ||
| | | | ||
| − | : | + | :The same rule for a liṭra of silk, which is 12 ounce. |
| − | |style="text-align:right;"|והוא הדין לליט' מן המשי שהוא י"ב אונק' עשה על זה הדרך | + | |style="text-align:right;"|והוא הדין לליט' מן המשי שהוא י"ב אונק‫' |
| + | |- | ||
| + | | | ||
| + | :Do this way. | ||
| + | |style="text-align:right;"|עשה על זה הדרך | ||
|- | |- | ||
| | | | ||
| Line 4,839: | Line 6,252: | ||
|- | |- | ||
| | | | ||
| − | : | + | :Take 20 dinar for each liṭra; and 1 and a fifth pešuṭim for each dinar |
| − | + | :<math>\scriptstyle{\color{OliveGreen}{X=\left[\left(20\sdot111\right)+7\right]\sdot\left(1+\frac{1}{5}\right)}}</math> | |
| − | + | |style="text-align:right;"|תפוש מכל ליט' כ' די' ומכל די' א' פשוט וחומש | |
| − | |style="text-align:right;"|תפוש מכל ליט' כ' די | + | |- |
| − | ומכל די' א' פשוט וחומש | + | | |
| − | וכן תעשה מככר לעשרון ומעשרון לאחד | + | :Do the same from a kikkar to tithes and from a tithe to units. |
| + | |style="text-align:right;"|וכן תעשה מככר לעשרון ומעשרון לאחד | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:pepper|629|0PNc}} | + | *{{#annot:pepper|629|0PNc}}If someone says: one ounce of pepper is sold for 7 pešiṭim. |
:How much is one kikkar worth? | :How much is one kikkar worth? | ||
:<math>\scriptstyle\frac{7}{20}=\frac{X}{100}</math> | :<math>\scriptstyle\frac{7}{20}=\frac{X}{100}</math> | ||
| − | |style="text-align:right;"|אם יאמר אדם האונקיא של פלפל נמכרה ז' פשיטי‫'<br> | + | |style="text-align:right;"|<big>אם יאמר אדם האונקיא</big> של פלפל נמכרה ז' פשיטי‫'<br> |
כמה יבא הכיכר{{#annotend:0PNc}} | כמה יבא הכיכר{{#annotend:0PNc}} | ||
|- | |- | ||
| | | | ||
| − | : | + | :Take 5 liṭra for each pašuṭ. |
| − | + | :<math>\scriptstyle{\color{OliveGreen}{X=7\sdot5}}</math> | |
|style="text-align:right;"|תפוש מכל פשוט ה' ליטרי' וכן כלם | |style="text-align:right;"|תפוש מכל פשוט ה' ליטרי' וכן כלם | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:pepper|629|S0eF}} | + | *{{#annot:pepper|629|S0eF}}If it is said: one ounce of pepper is sold for 8 pešiṭim. |
:How much are 1000 [liṭra] worth? | :How much are 1000 [liṭra] worth? | ||
:<math>\scriptstyle\frac{8}{20}=\frac{X}{1000}</math> | :<math>\scriptstyle\frac{8}{20}=\frac{X}{1000}</math> | ||
| Line 4,866: | Line 6,280: | ||
|- | |- | ||
| | | | ||
| − | : | + | :Take 50 liṭra for each pašuṭ. |
|style="text-align:right;"|תפוש מכל פשוט נ' ליטרי‫' | |style="text-align:right;"|תפוש מכל פשוט נ' ליטרי‫' | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{X=8\sdot50=400}}</math> | + | :Say: 8 times 50 liṭra are 400 liṭra. |
| + | :<math>\scriptstyle{\color{blue}{X=8\sdot50=400}}</math> | ||
|style="text-align:right;"|ואמור ח' פעמי' נ' ליט' הם ת' ליטרי‫' | |style="text-align:right;"|ואמור ח' פעמי' נ' ליט' הם ת' ליטרי‫' | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:silver|629|fjUl}} | + | *{{#annot:silver|629|fjUl}}If someone says: one marco of silver, which is 8 ounce, is worth 7 liṭra and 5 dinar. |
:How much is one ounce worth? | :How much is one ounce worth? | ||
:<math>\scriptstyle\frac{12\sdot\left[\left(20\sdot7\right)+5\right]}{8}=\frac{X}{1}</math> | :<math>\scriptstyle\frac{12\sdot\left[\left(20\sdot7\right)+5\right]}{8}=\frac{X}{1}</math> | ||
| − | |style="text-align:right;"|אם יאמר אדם המארקו של כסף שהוא ח' אונקי' שוה ז' ליטרי' וה' דינרי‫'<br> | + | |style="text-align:right;"|<big>אם יאמר אדם המארקו של כסף</big> שהוא ח' אונקי' שוה ז' ליטרי' וח' <sup>וה'</sup> דינרי‫'<br> |
כמה בא האונקי‫'{{#annotend:fjUl}} | כמה בא האונקי‫'{{#annotend:fjUl}} | ||
|- | |- | ||
| | | | ||
| − | : | + | :Take for each liṭra 20 dinar; and for each dinar one pašuṭ and a half. |
| − | + | :<math>\scriptstyle{\color{OliveGreen}{X=\left[\left(20\sdot7\right)+5\right]\sdot\left(1+\frac{1}{2}\right)}}</math> | |
| − | |style="text-align:right;"|תפוש מכל ליטרא כ' דינרי | + | |style="text-align:right;"|תפוש מכל ליטרא כ' דינרי' וחצי ומכל דינר א' פשוט וחצי |
| − | ומכל דינר א' פשוט וחצי | ||
| − | |||
|- | |- | ||
| | | | ||
| − | *{{#annot:liṭra|629|BPvK}} | + | :Do this way for all. |
| + | |style="text-align:right;"|וכן יבא ועל זה הדרך תעשה כלם | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:liṭra|629|BPvK}}If it is said: one liṭra is worth 5 dinar. | ||
:You want to know how much 1000 [liṭra] are worth | :You want to know how much 1000 [liṭra] are worth | ||
:<math>\scriptstyle\frac{5}{1}=\frac{20X}{1000}</math> | :<math>\scriptstyle\frac{5}{1}=\frac{20X}{1000}</math> | ||
| Line 4,895: | Line 6,312: | ||
|- | |- | ||
| | | | ||
| − | : | + | :Take 50 liṭra for each dinar. |
| − | + | :<math>\scriptstyle{\color{OliveGreen}{X=5\sdot50}}</math> | |
|style="text-align:right;"|תפוש מכל דינר נ' ליט‫' | |style="text-align:right;"|תפוש מכל דינר נ' ליט‫' | ||
|- | |- | ||
| | | | ||
| − | :If there are pešiṭim there | + | :If there are pešiṭim there: |
:<math>\scriptstyle\frac{5}{1}=\frac{12\sdot20\sdot X}{1000}</math> | :<math>\scriptstyle\frac{5}{1}=\frac{12\sdot20\sdot X}{1000}</math> | ||
|style="text-align:right;"|ואם היו שם פשיטי‫' | |style="text-align:right;"|ואם היו שם פשיטי‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | :Take 4 liṭra and [40] pešiṭim for each 1 pašuṭ. |
| − | + | :<math>\scriptstyle{\color{OliveGreen}{X=\left(4+\frac{40}{20\sdot12}\right)\sdot5}}</math> | |
| − | |style="text-align:right;"|תפוש מכל פשוט א' ד' ליטרי' | + | |style="text-align:right;"|תפוש מכל פשוט א' ד' ליטרי' וי' פשיטי‫' |
| + | |- | ||
| + | | | ||
| + | :Do the same for any number you want. | ||
| + | |style="text-align:right;"|וכן תעשה לכל חשבון שתרצה | ||
|- | |- | ||
| | | | ||
| Line 4,917: | Line 6,338: | ||
|- | |- | ||
| | | | ||
| − | :: | + | :Say: 6 times 150 is 900. |
| − | + | |style="text-align:right;"|אמור ו' פעמי' ק"נ תת"ק | |
| − | |style="text-align:right;"|אמור ו' פעמי' ק"נ תת"ק | ||
| − | |||
|- | |- | ||
| − | |1 | + | | |
| − | |style="text-align:right;"| | + | :Take 1 pašuṭ for every 25; the result is 36 pešiṭim and so the liṭra is worth. |
| + | :<math>\scriptstyle{\color{blue}{X=\frac{6\sdot150}{25\sdot\left(12\sdot20\right)}=\frac{900}{25\sdot\left(12\sdot20\right)}=\frac{36}{20\sdot12}}}</math> | ||
| + | |style="text-align:right;"|ותפוש מכל כ"ה‫<ref>כ"ה: MS Ithaca אלף</ref> א' פשוט שיבואו ל"ו פשיטי' וכן יבא הליט‫' | ||
|- | |- | ||
| − | | | + | |The ounce of gold is 30 terri. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<big>האונקיא של זהב</big> הוא ל' טרי |
|- | |- | ||
| − | | | + | |The terri is [5] groni. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והטרי הם גארוני |
| + | |- | ||
| + | |The groni is 4 grani. | ||
| + | |style="text-align:right;"|והגרוני הם ד' גראניו | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:gold|629|bcgS}} | + | *{{#annot:gold|629|bcgS}}If someone says: one ounce of gold is sold for 5 liṭra and 15 dinar. |
:How much is one terri worth? | :How much is one terri worth? | ||
:<math>\scriptstyle\frac{\left[5\sdot\left(12\sdot20\right)\right]+\left(15\sdot12\right)}{30}=\frac{X}{1}</math> | :<math>\scriptstyle\frac{\left[5\sdot\left(12\sdot20\right)\right]+\left(15\sdot12\right)}{30}=\frac{X}{1}</math> | ||
| − | |style="text-align:right;"|ואם יאמר אדם האונקיא של זהב נמכרה ה' ליטרי' וט"ו דינרי‫'<br> | + | |style="text-align:right;"|<big>ואם יאמר אדם האונקיא של</big> זהב נמכרה ה' ליטרי' וט"ו דינרי‫'<br> |
כמה יבא הטרי‫'{{#annotend:bcgS}} | כמה יבא הטרי‫'{{#annotend:bcgS}} | ||
|- | |- | ||
| | | | ||
| − | : | + | :Take 8 pešiṭim for each liṭra, and 2-fifths for each dinar. |
| − | + | :<math>\scriptstyle{\color{OliveGreen}{X=\left(5\sdot8\right)+\left(\frac{2}{5}\sdot15\right)}}</math> | |
| − | |style="text-align:right;"|תפוש מכל ליטר' ח"פ ומכל דינר ב' חמשיות | + | |style="text-align:right;"|תפוש מכל ליטר' ח"פ ומכל דינר ב' חמשיות‫<ref>ב': MS Ithaca ח'</ref> |
|- | |- | ||
| | | | ||
| − | *{{#annot:gold|629|SL63}} | + | :The same for any number you want. |
| − | :How much is one | + | |style="text-align:right;"|וכן לכל חשבון שתרצה |
| + | |- | ||
| + | | | ||
| + | *{{#annot:gold|629|SL63}}If it is said: one ounce of gold is worth 6 liṭra. | ||
| + | :How much is one garoni worth? | ||
:<math>\scriptstyle\frac{6\sdot\left(12\sdot20\right)}{30\sdot5}=\frac{X}{1}</math> | :<math>\scriptstyle\frac{6\sdot\left(12\sdot20\right)}{30\sdot5}=\frac{X}{1}</math> | ||
|style="text-align:right;"|ואם יאמר האונקי' של זהב שוה ו' ליטרי‫'<br> | |style="text-align:right;"|ואם יאמר האונקי' של זהב שוה ו' ליטרי‫'<br> | ||
| − | כמה יבא | + | כמה יבא הגרונא{{#annotend:SL63}} |
| + | |- | ||
| + | | | ||
| + | :Take 1 pašuṭ and 3-fifths for each liṭra. | ||
| + | :<math>\scriptstyle{\color{OliveGreen}{X=6\sdot\left(1+\frac{3}{5}\right)}}</math> | ||
| + | |style="text-align:right;"|תפוש מכל ליטר' א' פשיט וג' חמישיות | ||
|- | |- | ||
| | | | ||
| − | :: | + | :If there are dinar there: |
| − | + | |style="text-align:right;"|ואם יהיו שם דינרי‫' | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | : | + | :Take 3 parts of 25 for each dinar. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|‫<ref>43v</ref>תפוש מכל דינר ג' חלקי' מכ"ה |
|- | |- | ||
| | | | ||
| − | :How much is one grani worth? | + | :If it is said: How much is one grani worth? |
:<math>\scriptstyle\frac{6\sdot\left(12\sdot20\right)}{30\sdot5\sdot4}=\frac{X}{1}</math> | :<math>\scriptstyle\frac{6\sdot\left(12\sdot20\right)}{30\sdot5\sdot4}=\frac{X}{1}</math> | ||
| − | |style="text-align:right;"|ואם יאמר כמה יבא | + | |style="text-align:right;"|ואם יאמר כמה יבא הגראנו‫' |
|- | |- | ||
| | | | ||
| − | : | + | :Take 2-fifths for each liṭra. |
|style="text-align:right;"|תפוש מכל ליטר' ב' חומשי | |style="text-align:right;"|תפוש מכל ליטר' ב' חומשי | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{X=6\sdot\frac{2}{5}=2+\frac{2}{5}}}</math> | + | :So, at one ounce for 6 liṭra, the grano is worth 2 pešiṭim and 2-fifths. |
| − | |style="text-align:right;"|הרי שיבא הגראנו לחשבון ו' ליטרין האונקי' ב' פשיטי וב' חמישיות | + | :<math>\scriptstyle{\color{blue}{X=6\sdot\frac{2}{5}=2+\frac{2}{5}}}</math> |
| + | |style="text-align:right;"|הרי שיבא הגראנו לחשבון <s>ב' חומשי'</s> ו' ליטרין האונקי' ב' פשיטי' וב' חמישיות | ||
| + | |- | ||
| + | | | ||
| + | :If there are dinar there: | ||
| + | |style="text-align:right;"|ואם יהיו שם דינרי‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | :Take one part of fifty for each dinar. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|תפוש מכל דינר א' חלק מחמשים |
|- | |- | ||
| | | | ||
| − | *{{#annot:ounce|629|dwJe}}One terri is worth 28 pešiṭim. | + | *{{#annot:ounce|629|dwJe}}If it is said: One terri is worth 28 pešiṭim. |
:How much is one ounce worth? | :How much is one ounce worth? | ||
:<math>\scriptstyle\frac{28}{1}=\frac{X}{30}</math> | :<math>\scriptstyle\frac{28}{1}=\frac{X}{30}</math> | ||
| − | |style="text-align:right;"|ואם יאמר הטרי שוה כ"ח פשיטי‫'<br> | + | |style="text-align:right;"|ואם יאמר הטרי שוה <s>כ</s> כ"ח פשיטי‫'<br> |
כמה יבא האוקי‫'{{#annotend:dwJe}} | כמה יבא האוקי‫'{{#annotend:dwJe}} | ||
|- | |- | ||
| | | | ||
| − | : | + | :Take 30 dinar for each dinar; and 30 pešiṭim for each pašuṭ. |
| − | + | :<math>\scriptstyle{\color{OliveGreen}{X=28\sdot30}}</math> | |
|style="text-align:right;"|תפוש מכל דינר ל' דינרי' ומכל פשוט ל' פשיטי‫' | |style="text-align:right;"|תפוש מכל דינר ל' דינרי' ומכל פשוט ל' פשיטי‫' | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | === Guessing Problems === | + | === <span style=color:green>Guessing Problems</span> === |
| | | | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:coins|667|qSYo}} | + | *{{#annot:three coins|667|qSYo}}Give three different coins to three people: one of gold, one of silver, and one of copper, and they should divide them between them without your knowledge. |
:You can guess [who has each coin] by giving one of them one coin, the second two [coins], and the third four [coins]. | :You can guess [who has each coin] by giving one of them one coin, the second two [coins], and the third four [coins]. | ||
:Then tell them that the one who has the gold should multiply [the number of] coins you gave him recently by 4. | :Then tell them that the one who has the gold should multiply [the number of] coins you gave him recently by 4. | ||
| Line 5,005: | Line 6,440: | ||
:Ask them what is the remainder. | :Ask them what is the remainder. | ||
:[The answer] will be known according to the [results]: 4, 6, 2, 5, 1, 3 | :[The answer] will be known according to the [results]: 4, 6, 2, 5, 1, 3 | ||
| − | |style="text-align:right;"|תן לג' אנשים ג' מטבעות שונות הא' מזהב וא' מכסף וא' מנחשת ויחלקום ביניהם בלתי ידיעתך<br> | + | |style="text-align:right;"|<big>תן לג' אנשים ג' מטבעות שונות</big> הא' מזהב וא' מכסף וא' מנחשת ויחלקום ביניהם בלתי ידיעתך<br> |
וזה תוכל לדעת שתתן לאחד מהם א' מטבע ולשני ב' ולשליש ד‫'<br> | וזה תוכל לדעת שתתן לאחד מהם א' מטבע ולשני ב' ולשליש ד‫'<br> | ||
ואמור להם שאותו שיש לו הזהב יכפול ד' פעמי' המטבעות שנתת לו באחרונה<br> | ואמור להם שאותו שיש לו הזהב יכפול ד' פעמי' המטבעות שנתת לו באחרונה<br> | ||
| − | ובעל הכסף יכפול ה' פעמים המטבעות שנתת לו באחרונה<br> | + | ובעל הכסף יכפול ה'‫<ref>ה': MS Ithaca ב'</ref> פעמים המטבעות שנתת לו באחרונה<br> |
ובעל הנחושת יכפול המטבעות שנתת לו באחרונה<br> | ובעל הנחושת יכפול המטבעות שנתת לו באחרונה<br> | ||
ויקבצו הכל וישליכו ז"ז<br> | ויקבצו הכל וישליכו ז"ז<br> | ||
ושאל הנשאר<br> | ושאל הנשאר<br> | ||
| − | וזה יודע על פי ד'ו'ב' ה'א'ג‫'{{#annotend:qSYo}} | + | וזה יודע על פי <big>ד'ו'ב' ה'א'ג‫'</big>{{#annotend:qSYo}} |
| + | |- | ||
| + | | | ||
| + | :Explanation: | ||
| + | |style="text-align:right;"|וביאור זה | ||
|- | |- | ||
| | | | ||
| − | : | + | :If they are left with 4, the order is: gold, silver, copper. |
| − | + | :<math>\scriptstyle{\color{OliveGreen}{\left[\left(4\sdot1\right)+\left(3\sdot2\right)+\left(2\sdot4\right)\right]mod7=4}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אם נשאר בידם ד' יהיה הסדר זהב כסף ונחושת |
|- | |- | ||
| | | | ||
| − | :[( | + | :If they are left with 6, the [order] is: gold, copper, silver. |
| + | :<math>\scriptstyle{\color{OliveGreen}{\left[\left(4\sdot1\right)+\left(2\sdot2\right)+\left(3\sdot4\right)\right]mod7=6}}</math> | ||
|style="text-align:right;"|ואם נשאר בידם ו' יהיה הנשאר יהיה הנשאר זהב ונחושת כסף | |style="text-align:right;"|ואם נשאר בידם ו' יהיה הנשאר יהיה הנשאר זהב ונחושת כסף | ||
|- | |- | ||
| | | | ||
| − | :[( | + | :If they are left with 2, the order is: silver, copper, gold. |
| − | |style="text-align:right;"|ואם נשאר בידם ב' יהיה הכסף כסף נחושת זהב | + | :<math>\scriptstyle{\color{OliveGreen}{\left[\left(3\sdot1\right)+\left(2\sdot2\right)+\left(4\sdot4\right)\right]mod7=2}}</math> |
| + | |style="text-align:right;"|ואם נשאר בידם ב' יהיה הסדר‫<ref>הסדר: MS Ithaca הכסף</ref> כסף נחושת זהב | ||
|- | |- | ||
| | | | ||
| − | :[( | + | :If they are left with 5, the order is: silver, gold, copper. |
| + | :<math>\scriptstyle{\color{OliveGreen}{\left[\left(3\sdot1\right)+\left(4\sdot2\right)+\left(2\sdot4\right)\right]mod7=5}}</math> | ||
|style="text-align:right;"|ואם נשאר בידם ה' יהיה הסדר כסף זהב נחושת | |style="text-align:right;"|ואם נשאר בידם ה' יהיה הסדר כסף זהב נחושת | ||
|- | |- | ||
| | | | ||
| − | :[( | + | :If they are left with 1, the order is: copper, gold, silver. |
| − | |style="text-align:right;"|ואם נשאר בידם א' יהיה הסדר פ נחשת זהב כסף | + | :<math>\scriptstyle{\color{OliveGreen}{\left[\left(2\sdot1\right)+\left(4\sdot2\right)+\left(3\sdot4\right)\right]mod7=1}}</math> |
| + | |style="text-align:right;"|ואם נשאר בידם א' יהיה הסדר <s>פ</s> נחשת זהב כסף | ||
|- | |- | ||
| | | | ||
| − | :[( | + | :If they are left with 3, the order is: copper, silver, gold. |
| + | :<math>\scriptstyle{\color{OliveGreen}{\left[\left(2\sdot1\right)+\left(3\sdot2\right)+\left(4\sdot4\right)\right]mod7=3}}</math> | ||
|style="text-align:right;"|ואם נשאר בידם ג' יהיה הסדר נחושת כסף זהב | |style="text-align:right;"|ואם נשאר בידם ג' יהיה הסדר נחושת כסף זהב | ||
|- | |- | ||
| Line 5,048: | Line 6,492: | ||
:Then sum up [the results] and cast out 105 repeatedly. | :Then sum up [the results] and cast out 105 repeatedly. | ||
:The remainder will be the number he chose. | :The remainder will be the number he chose. | ||
| − | + | |style="text-align:right;"|<big>תאמר לאיש שיחשוב אך לא פחות</big> מז' וישליכם ג' ג' ג' ושאל הנשאר<br> | |
| − | |style="text-align:right;"|תאמר לאיש שיחשוב אך לא פחות מז' וישליכם ג' ג' ג' ושאל הנשאר<br> | ||
אחר כן ישליך מה שחשב ה' ה' ושאל הנשאר<br> | אחר כן ישליך מה שחשב ה' ה' ושאל הנשאר<br> | ||
אחר זה ישליך מה שחשב ז' ז' ושאל הנשאר<br> | אחר זה ישליך מה שחשב ז' ז' ושאל הנשאר<br> | ||
| − | ומכל מה שנשאר אחר שהשליכם ג' ג' תחשוב לכל אחד הנ[ות]ר מן הג' ע‫'<br> | + | ומכל מה שנשאר אחר שהשליכם ג' ג' תחשוב‫<ref>תחשוב: MS Ithaca om.</ref> לכל אחד הנ[ות]ר מן הג' ע‫'<br> |
| − | ומכל מה שנשאר אחר שהשליכם ה'ה' תחשוב לכל אחד כ"א<br> | + | ומכל מה שנשאר אחר שהשליכם ה'ה' תחשוב לכל אחד‫<ref>ומכל... לכל אחד: MS Ithaca ולכל הנשאר מן הה'</ref> כ"א<br> |
| − | ומכל | + | ומכל הנשאר אחר שהשליכם ז' ז' קח לכל אחד ט"ו<br> |
| − | וצרפם והשלך אותם ק"ה והנותר יהיה מה שחשב והסימן ג"ע הכ"א זט"ו{{#annotend:Quwt}} | + | וצרפם והשלך אותם ק"ה והנותר יהיה מה שחשב <big>והסימן ג"ע הכ"א זט"ו</big>{{#annotend:Quwt}} |
| + | |- | ||
| + | | colspan="2"| | ||
| + | :<math>\scriptstyle\left[\left[\left(x\, mod3\right)\sdot70\right]+\left[\left(x\, mod5\right)\sdot21\right]+\left[\left(x\, mod7\right)\sdot15\right]\right]mod105=x</math> | ||
|- | |- | ||
| | | | ||
| − | :* | + | :*If he chose [the number] 17 |
|style="text-align:right;"|דמיון זה כאלו חשב י"ז | |style="text-align:right;"|דמיון זה כאלו חשב י"ז | ||
|- | |- | ||
| | | | ||
| + | ::We tell him to cast out the threes; he is left with 2. | ||
| + | |style="text-align:right;"|אמרנו שישליכם ג'ג' וישאר בידו ב‫' | ||
| + | |- | ||
| + | | | ||
| + | ::We tell him to cast out the fives; he is left with 2. | ||
| + | |style="text-align:right;"|אחר כן אמרנו לו שישליכם ה'ה' וישאר בידו ב‫' | ||
| + | |- | ||
| + | | | ||
| + | ::We tell him to cast out the sevens; he is left with 3. | ||
| + | |style="text-align:right;"|אחר כן אמרנו לו שישליכם ז'ז' וישאר בידו ג‫' | ||
| + | |- | ||
| + | | | ||
| + | ::For what remains when cast out the threes, which is 2, think of each unit as 70; it is 140. | ||
| + | |style="text-align:right;"|ממה שנשאר כשהשליכם ג'ג' דהיין ב' תחשוב לכל אחד ע' ויהיו ק"מ | ||
| + | |- | ||
| + | | | ||
| + | ::For what remains when cast out the fives, which is 2, think of each unit as 21; it is 42. | ||
| + | |style="text-align:right;"|וממה שנשאר כשהשליכם ה'ה' דהיין ב' תחשוב לכל אחד כ"א ויהיו מ"ב | ||
| + | |- | ||
| + | | | ||
| + | ::For what remains when cast out the sevens, which is 3, think of each unit as 15; it is 45. | ||
| + | |style="text-align:right;"|ומה שנשאר כשהשליכם ז'ז' דהיין ג' תחשוב לכל אחד ט"ו ויהיו מ"ה | ||
| + | |- | ||
| + | | | ||
| + | ::Sum it all up; it is 227. | ||
| + | |style="text-align:right;"|קבץ הכל ויהיו רכ"ז | ||
| + | |- | ||
| + | | | ||
| + | ::Cast out the 105s; you are left with 17, as [the number] your friend chose. | ||
| + | |style="text-align:right;"|השליכם ק"ה ק"ה ישארו בידך י"ז כמו שחשב חברך‫<ref>דמיון זה... כמו שחשב חברך: according to MS Verona; MS Ithaca om.</ref> | ||
| + | |- | ||
| + | | colspan="2"| | ||
::<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle x&\scriptstyle=\left[\left[\left(17\, mod3\right)\sdot70\right]+\left[\left(17\, mod5\right)\sdot21\right]+\left[\left(17\, mod7\right)\sdot15\right]\right]mod105\\&\scriptstyle=\left[\left(2\sdot70\right)+\left(2\sdot21\right)+\left(3\sdot15\right)\right]mod105\\&\scriptstyle=\left(140+42+45\right)mod105\\&\scriptstyle=227mod105=17\\\end{align}}}</math> | ::<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle x&\scriptstyle=\left[\left[\left(17\, mod3\right)\sdot70\right]+\left[\left(17\, mod5\right)\sdot21\right]+\left[\left(17\, mod7\right)\sdot15\right]\right]mod105\\&\scriptstyle=\left[\left(2\sdot70\right)+\left(2\sdot21\right)+\left(3\sdot15\right)\right]mod105\\&\scriptstyle=\left(140+42+45\right)mod105\\&\scriptstyle=227mod105=17\\\end{align}}}</math> | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | === Divide a Quantity Problem === | + | === <span style=color:green>Divide a Quantity Problem</span> === |
| | | | ||
| Line 5,087: | Line 6,555: | ||
:How should they share the [five pešiṭim]? | :How should they share the [five pešiṭim]? | ||
:<math>\scriptstyle\left(2-\frac{5}{3}\right)X+\left(3-\frac{5}{3}\right)X=5</math> | :<math>\scriptstyle\left(2-\frac{5}{3}\right)X+\left(3-\frac{5}{3}\right)X=5</math> | ||
| − | |style="text-align:right;"|שני אנשים היו יושבים לאכול<br> | + | |style="text-align:right;"|‫<ref>44r</ref><big>שני אנשים היו יושבים לאכול</big><br> |
| − | לאחד היו ג' לחמים ולשני ב' לחמים<br> | + | <big>לאחד</big> היו <s>ב'</s> ג'‫<ref>ג': MS Ithaca marg.</ref> לחמים ולשני ב' לחמים<br> |
בא אדם אחד שלישי ואכל עמהם ואכלו בין שלשתם אילו הה' לחמים<br> | בא אדם אחד שלישי ואכל עמהם ואכלו בין שלשתם אילו הה' לחמים<br> | ||
| − | + | לאחר שאכלו נתן אותו השליש שאכל עמהם ה' פשיטי שיחלקום ביניהם<br> | |
היאך חלקו ביניהם{{#annotend:FQns}} | היאך חלקו ביניהם{{#annotend:FQns}} | ||
|- | |- | ||
| | | | ||
| − | ::each | + | :Say: How much of the bread did each of them eat? 1 loaf of bread and 2-thirds. |
| + | :<math>\scriptstyle{\color{blue}{\frac{5}{3}=1+\frac{2}{3}}}</math> | ||
|style="text-align:right;"|אמור כמה חלק מן הלחם אכל כל אחד מהם א' לחם וב' שלישיות לחם | |style="text-align:right;"|אמור כמה חלק מן הלחם אכל כל אחד מהם א' לחם וב' שלישיות לחם | ||
|- | |- | ||
| | | | ||
| − | : | + | :Then, the one who had 2 loaves of bread and ate 1 loaf of bread and 2-thirds, lost a third of a loaf. |
| + | :<math>\scriptstyle{\color{blue}{2-\left(1+\frac{2}{3}\right)=\frac{1}{3}}}</math> | ||
|style="text-align:right;"|אם כן אותו שהיו לו ב' לחמים ואכל א' לחם וב' שלישיות לא הפסיד כי אם שליש לחם | |style="text-align:right;"|אם כן אותו שהיו לו ב' לחמים ואכל א' לחם וב' שלישיות לא הפסיד כי אם שליש לחם | ||
|- | |- | ||
| | | | ||
| − | : | + | :The one who had 3 loaves of bread and ate 1 loaf of bread and 2-thirds, lost 1 loaf of bread and a third, which is 4-thirds of a loaf. |
| − | |style="text-align:right;"|ואותו שהיו לו ג' לחמים ואכל א' לחם וב' שלישיות הפסיד א' ושליש | + | :<math>\scriptstyle{\color{blue}{3-\left(1+\frac{2}{3}\right)=1+\frac{1}{3}=\frac{4}{3}}}</math> |
| + | |style="text-align:right;"|ואותו שהיו לו ג' לחמים ואכל א' לחם וב' שלישיות <sup><s>לא</s></sup> הפסיד <s>כי אם שליש</s> <sup>א'</sup> לחם ושליש שהם ד' שלישיו' לחם | ||
|- | |- | ||
| | | | ||
| − | : | + | :So, the one who had 2 loaves of bread will receive 1 pašuṭ, because he lost a third of a loaf. |
| + | :<math>\scriptstyle{\color{OliveGreen}{a_2=1}}</math> | ||
|style="text-align:right;"|אם כן אותו שיש לו ב' לחמים יקבל א' פשוט בעבור שהפסיד שליש לחם | |style="text-align:right;"|אם כן אותו שיש לו ב' לחמים יקבל א' פשוט בעבור שהפסיד שליש לחם | ||
|- | |- | ||
| | | | ||
| − | : | + | :The one who had 3 loaves of bread will receive 4 pešiṭim, because he lost 4-thirds of a loaf. |
| + | :<math>\scriptstyle{\color{OliveGreen}{a_3=4}}</math> | ||
|style="text-align:right;"|ואותו שיש לו ג' לחמים יקבל ד' פשיטי' כי הפסיד ד' שלישי לחם | |style="text-align:right;"|ואותו שיש לו ג' לחמים יקבל ד' פשיטי' כי הפסיד ד' שלישי לחם | ||
|- | |- | ||
| | | | ||
| − | === Motion Problem - To and From === | + | === <span style=color:green>Motion Problem - To and From</span> === |
| | | | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:ant climbing | + | *{{#annot:ant climbing|659|0jPu}}A tower is 20 cubits tall. |
:An ant wants to climb up. | :An ant wants to climb up. | ||
:Every day it climbs up one third of a cubit and every night it goes down a quarter of a cubit. | :Every day it climbs up one third of a cubit and every night it goes down a quarter of a cubit. | ||
:How much is its progress each day and in how many days it will reach to the top?<br> | :How much is its progress each day and in how many days it will reach to the top?<br> | ||
:<math>\scriptstyle\frac{1}{3}X-\frac{1}{4}X=20</math> | :<math>\scriptstyle\frac{1}{3}X-\frac{1}{4}X=20</math> | ||
| − | |style="text-align:right;"|מגדל שהוא גבוה עשרים אמה<br> | + | |style="text-align:right;"|<big>מגדל שהוא גבוה עשרים אמה</big><br> |
ונמלה אחת רוצה לעלות למעלה<br> | ונמלה אחת רוצה לעלות למעלה<br> | ||
ובכל יום עולה שליש אמה ובכל לילה יורדת רביע אמה<br> | ובכל יום עולה שליש אמה ובכל לילה יורדת רביע אמה<br> | ||
| Line 5,131: | Line 6,604: | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\frac{1}{3}-\frac{1}{4}=\frac{1}{12}}}</math> | + | :First, say: how much greater is the third than the quarter? 1 part of 12. |
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{3}-\frac{1}{4}=\frac{1}{12}}}</math> | ||
|style="text-align:right;"|אמור תחלה כמה הוא יותר השליש מן הרביע א' חלק מי"ב | |style="text-align:right;"|אמור תחלה כמה הוא יותר השליש מן הרביע א' חלק מי"ב | ||
|- | |- | ||
| | | | ||
| − | : | + | :So, it climbs up one cubit in 12 days. |
| − | |style="text-align:right;"|הרי שי"ב ימים היא עולה א' אמה | + | |style="text-align:right;"|הרי שי"ב ימים היא<ref>שי"ב ימים היא: MS Ithaca twice</ref> עולה א' אמה |
| + | |- | ||
| + | | | ||
| + | :Since the tower is 20 cubits tall, say: 12 times 20 is 240. | ||
| + | :<math>\scriptstyle{\color{blue}{x=12\sdot20=240}}</math> | ||
| + | |style="text-align:right;"|ובעבור שהמגדל הוא גבוה כ' אמה אמור י"ב פעמים כ' הם ר"מ | ||
|- | |- | ||
| | | | ||
| − | : | + | :Therefore, it will reach the top in 240 days. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|הרי שבר"מ ימים תגיע בראש המגדל |
| − | הרי שבר"מ ימים | ||
|- | |- | ||
| | | | ||
| + | :And so on. | ||
|style="text-align:right;"|וכן כלם | |style="text-align:right;"|וכן כלם | ||
|- | |- | ||
| | | | ||
| − | === Find a Quantity Problem - First from Last === | + | === <span style=color:green>Find a Quantity Problem - First from Last</span> === |
| | | | ||
| Line 5,154: | Line 6,633: | ||
| | | | ||
*{{#annot:money in purse|651|Y4nv}}You have some money in your purse. | *{{#annot:money in purse|651|Y4nv}}You have some money in your purse. | ||
| − | :You take a third, a quarter, and a fifth of it and their sum is | + | :You take a third, a quarter, and a fifth of it and their sum is 9 pešiṭim. |
:How much remains? | :How much remains? | ||
| − | :<math>\scriptstyle X-\frac{1}{3}X+\frac{1}{4}X+\frac{1}{5}X=X-9</math> | + | :<math>\scriptstyle X-\left(\frac{1}{3}X+\frac{1}{4}X+\frac{1}{5}X\right)=X-9</math> |
| − | |style="text-align:right;"|הרי שיש לך מעות בכיס<br> | + | |style="text-align:right;"|<big>הרי שיש לך מעות בכיס</big><br> |
והוצאת מהם השליש והרביע והחומש והם ט' פשיטים<br> | והוצאת מהם השליש והרביע והחומש והם ט' פשיטים<br> | ||
| − | כמה יהיו הנשארים{{#annotend:Y4nv}} | + | כמה יהיו הנשארים{{#annotend:Y4nv}}‫<ref>והם ט‫': MS ithaca twice</ref> |
|- | |- | ||
| | | | ||
| − | :: | + | :Say: a third, a quarter and a fifth are found in 60. |
| − | |style="text-align:right;"|אמור שליש ורביע וחומש ימצאו בס' | + | |style="text-align:right;"|אמור שליש ורביע וחומש ימצאו בס‫' |
|- | |- | ||
| | | | ||
| − | ::<span style=color: | + | :The third is 20; the quarter is 15; the fifth is 12; their total is 47. |
| + | :<math>\scriptstyle{\color{blue}{\left(\frac{1}{3}\sdot60\right)+\left(\frac{1}{4}\sdot60\right)+\left(\frac{1}{5}\sdot60\right)=20+15+12=47}}</math> | ||
| + | |style="text-align:right;"|השליש הוא כ' הרביע הוא ט"ו החומש י"ב וכללם מ"ז | ||
| + | |- | ||
| + | | | ||
| + | :<span style=color:green>'''False Position:'''</span> So, the third, the quarter, and the fifth, which are 9 pešiṭim, are 47 parts of 60. | ||
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{3}+\frac{1}{4}+\frac{1}{5}=\frac{47}{60}}}</math> | ||
|style="text-align:right;"|הרי שהשליש והרביע והחומש שהם ט"פ הם מ"ז חלקים מס‫' | |style="text-align:right;"|הרי שהשליש והרביע והחומש שהם ט"פ הם מ"ז חלקים מס‫' | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\frac{60}{60}-\frac{47}{60}=\frac{13}{60}}}</math> | + | :We find that 13 parts of 60 are left in the purse. |
| + | :<math>\scriptstyle{\color{blue}{\frac{60}{60}-\frac{47}{60}=\frac{13}{60}}}</math> | ||
|style="text-align:right;"|נמצא שהנשאר בכיס הם י"ג חלקי' מס‫' | |style="text-align:right;"|נמצא שהנשאר בכיס הם י"ג חלקי' מס‫' | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> Say: if 47 parts of 60 are equal to 9 pešiṭim, how much are 13 parts of 60 equal to? | |
| + | :<math>\scriptstyle{\color{blue}{\frac{47}{60}:9=\frac{13}{60}:a}}</math> | ||
|style="text-align:right;"|ולכן אמור אם מ"ז חלקי' מס' שוי' ט"פ י"ג חלקי' מס' כמה שוים | |style="text-align:right;"|ולכן אמור אם מ"ז חלקי' מס' שוי' ט"פ י"ג חלקי' מס' כמה שוים | ||
|- | |- | ||
| | | | ||
| − | :: | + | :Say: 13 times 9 is 117. |
| − | |style="text-align:right;"|אמור י"ג פעמי' ט' קי | + | |style="text-align:right;"|אמור י"ג פעמי' ‫<ref>44v</ref>ט' קי"ז |
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :Divide it by 47; the result is 2 and 23 parts of 47. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{a=\frac{13\sdot9}{47}=\frac{117}{47}=2+\frac{23}{47}}}</math> |
| + | |style="text-align:right;"|חלקם במ"ז יבאו ב' וכ"ג חלקים ממ"ז | ||
|- | |- | ||
| | | | ||
| − | ::the money that remained: <math>\scriptstyle{\color{blue}{\left(11+\frac{23}{47}\right)-9=2+\frac{23}{47}}}</math> | + | :We find that the money that was in the purse is 11 pešiṭim and 23 parts of 47. |
| − | |style="text-align:right;"|ומה שהוציא היו ט' ומה שנשאר היו ב' וכ"ג חלקים ממ"ז וכן לכל חשבון שתרצה | + | :<math>\scriptstyle{\color{blue}{X=11+\frac{23}{47}}}</math> |
| + | |style="text-align:right;"|נמצא שהיו בתוך הכיס י"א פשו' וכ"ג חלקים ממ"ז‫<ref>נמצא שהיו... ממ"ז: MS Ithaca om.</ref> | ||
| + | |- | ||
| + | | | ||
| + | :9 [pešiṭim] were taken out and [the money] that remained is 2 pešiṭim and 23 parts of 47. | ||
| + | :<math>\scriptstyle{\color{blue}{\left(11+\frac{23}{47}\right)-9=2+\frac{23}{47}}}</math> | ||
| + | |style="text-align:right;"|ומה שהוציא היו ט' ומה שנשאר היו ב' וכ"ג חלקים ממ"ז | ||
| + | |- | ||
| + | | | ||
| + | :The same for any number you want. | ||
| + | |style="text-align:right;"|וכן לכל חשבון שתרצה | ||
|- | |- | ||
| | | | ||
| − | === Ordering Problems === | + | === <span style=color:green>Ordering Problems</span> === |
| | | | ||
| Line 5,201: | Line 6,698: | ||
:But he has only two jars – one with a maximum capacity of 3 cups and the other [with a maximum capacity of] 5 [cups]. | :But he has only two jars – one with a maximum capacity of 3 cups and the other [with a maximum capacity of] 5 [cups]. | ||
:How will he divide it into two? | :How will he divide it into two? | ||
| − | |style="text-align:right;"|אדם שיש לו צלוחית אחת | + | |style="text-align:right;"|<big>אדם שיש לו צלוחית אחת שהיא</big> מחזקת ח' כוסות יין<br> |
ורוצה לחלקו לב' אנשי' וליתן לכל אחד ד' מנות<br> | ורוצה לחלקו לב' אנשי' וליתן לכל אחד ד' מנות<br> | ||
אמנם אין לו רק ב' כלים שהאחד מהם מחזיק ג' והאחר מחזיק ה‫'<br> | אמנם אין לו רק ב' כלים שהאחד מהם מחזיק ג' והאחר מחזיק ה‫'<br> | ||
| − | היאך יעשה אותם לחלק זה היין לחצי{{#annotend:Dsq4}} | + | היאך יעשה אותם לחלק זה היין‫<ref>זה היין: MS Ithaca אותם</ref> לחצי{{#annotend:Dsq4}} |
|- | |- | ||
| | | | ||
| − | : | + | :He will fill the jar that holds 3 [cups] and pour [its content] into the jar that holds 5 [cups]. |
| − | + | |style="text-align:right;"|ימלא הכלי שמחזיק ג' וישי' אותם בכלי שמחזיק ה‫' | |
| − | |style="text-align:right;"|ימלא הכלי שמחזיק ג' | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>3 cups - medium jar<br>5 cups - large jar</span> | |
| − | + | | | |
| − | | | ||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :Then, he will fill again the jar that holds 3 [cups] and pour 2 [cups] from it into the jar that holds 5 [cups]. |
| − | + | |style="text-align:right;"|אחרי כן ימלא שנית הכלי שמחזיק ג' וישים מהם בכלי שמחזיק ה' ב‫' | |
| − | |style="text-align:right;"| | ||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :We find that the jar that holds 5 [cups] is full; the jar that holds 3 [cups] has 1 [cup]; and the jar that holds 8 [cups] has 2 [cups]. |
| − | + | |style="text-align:right;"|נמצא שהכלי שמחזיק ה' הוא מלא והכלי שמחזיק ג' יש שם א' והכלי שמחזיק ח' יש שם ב‫' | |
| − | |style="text-align:right;"| | ||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | *{{#annot:barrels of wine|628|SDsn}}A man has 12 barrels of wine: | + | :<span style=color:green>1 cup - small jar<br>5 cups - medium jar<br>2 cups - large jur</span> |
| − | :one barrel contains one measure; | + | | |
| + | |- | ||
| + | | | ||
| + | :Next, he will pour [the content] of the jar that holds 5 [cups], which is full, into the jug that holds 8 [cups]. | ||
| + | |style="text-align:right;"|ישים אחר כן הכלי שמחזיק ה' שהוא מלא לתוך הכלי שמחזיק ח‫' | ||
| + | |- | ||
| + | | | ||
| + | :We find that there are 7 [cups] in that jar. | ||
| + | |style="text-align:right;"|נמצא שיש באותו הכלי ז' כוסות | ||
| + | |- | ||
| + | | | ||
| + | :<span style=color:green>1 cup - small jar<br>7 cups - large jar</span> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :Then, he will pour the one cup that is in the jar that holds 3 [cups] into the jar that hold 5 [cups]. | ||
| + | |style="text-align:right;"|ישים אחרי כן אותו הכוס האחד שהוא בכלי שמחזיק ג' בתוך הכלי שמחזיק ה‫' | ||
| + | |- | ||
| + | | | ||
| + | :He will fill the jar that holds 3 [cups] and pour [its content] into the jar that holds 5 [cups] | ||
| + | |style="text-align:right;"|וימלא הכלי שמחזיק ג' וישים אותם בכלי שמחזיק ה‫' | ||
| + | |- | ||
| + | | | ||
| + | :So, there are 4 cups there and in the jar that holds 8 [cups], there are 4 cups left. | ||
| + | |style="text-align:right;"|הרי שיש שם ד' כוסות ובכלי שמחזיק ח' נשארו ד' כוסות | ||
| + | |- | ||
| + | | | ||
| + | :<span style=color:green>4 cups - medium jar<br>4 cups - large jar</span> | ||
| + | | | ||
| + | |- | ||
| + | | | ||
| + | :Thus, they are divided in half. | ||
| + | |style="text-align:right;"|הרי שהם נחלקים לחצי | ||
| + | |- | ||
| + | | | ||
| + | *{{#annot:barrels of wine|628|SDsn}}A man has 12 barrels of wine: | ||
| + | :one barrel contains one measure; | ||
:the second [contains] two [measures]; | :the second [contains] two [measures]; | ||
:the third [contains] three [measures]; | :the third [contains] three [measures]; | ||
:and so on until the twelve [barrel]. | :and so on until the twelve [barrel]. | ||
:He wants to divide [the barrels] between his three sons, so that each of them will have the same amount of wine and the same number of barrels. | :He wants to divide [the barrels] between his three sons, so that each of them will have the same amount of wine and the same number of barrels. | ||
| − | :How should he divide them so that each will have 4 barrels containing 26 measures of wine? | + | :How should he divide them so that each will have 4 barrels containing 26 measures of wine? |
| − | |style="text-align:right;"|אדם אחד שהיו לו י"ב חביות יין<br> | + | |style="text-align:right;"|‫<ref>This problem is missing in MS Ithaca; it appears in MS Verona, Cambridge</ref>אדם אחד שהיו לו י"ב חביות יין<br> |
הא' מחזקת א' משואי<br> | הא' מחזקת א' משואי<br> | ||
והשנית ב‫'<br> | והשנית ב‫'<br> | ||
| Line 5,248: | Line 6,772: | ||
|- | |- | ||
| | | | ||
| − | : | + | :He should divide them as follows: |
|style="text-align:right;"|יחלקם על זה הסדר כגון זה | |style="text-align:right;"|יחלקם על זה הסדר כגון זה | ||
|- | |- | ||
| | | | ||
| − | + | :{|class="wikitable" style="text-align:center;" | |
|- | |- | ||
| | | | ||
| Line 5,279: | Line 6,803: | ||
|- | |- | ||
|} | |} | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | === Find a Quantity Problem - Whole from Parts === | + | === <span style=color:green>Find a Quantity Problem - Whole from Parts</span> === |
| | | | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:fish|650|IjMQ}} | + | *{{#annot:fish|650|IjMQ}}You have a fish - its head and tail were cut off. |
:The body weighs 10 liṭra. | :The body weighs 10 liṭra. | ||
:The head weighs one third and a quarter of the whole fish. | :The head weighs one third and a quarter of the whole fish. | ||
| Line 5,295: | Line 6,817: | ||
:How much does the whole fish weigh? | :How much does the whole fish weigh? | ||
:<math>\scriptstyle\frac{1}{3}X+\frac{1}{4}X+\frac{1}{5}X+\frac{1}{6}X+10=X</math> | :<math>\scriptstyle\frac{1}{3}X+\frac{1}{4}X+\frac{1}{5}X+\frac{1}{6}X+10=X</math> | ||
| − | |style="text-align:right;"|הרי שיש לפניך דג ונחתך ראשו וזנבו<br> | + | |style="text-align:right;"|‫<ref>MS Ithaca om.</ref>הרי שיש לפניך דג ונחתך ראשו וזנבו<br> |
והגוף שוקל י' ליט‫'<br> | והגוף שוקל י' ליט‫'<br> | ||
והראש שוקל השליש והרביע מכל הדג<br> | והראש שוקל השליש והרביע מכל הדג<br> | ||
| Line 5,302: | Line 6,824: | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''False Position:'''</span> First say: a third, a quarter, a fifth, and a sixth are found in 60; they are 20, 15, 12, and 10. | |
| − | |style="text-align:right;"|אמור תחלה שליש ורביע וחומש ושתות ימצאו בס‫' | + | |style="text-align:right;"|אמור תחלה שליש ורביע וחומש ושתות ימצאו בס' שהם כ' וט"ו וי"ב וי‫' |
|- | |- | ||
| | | | ||
| − | : | + | :Their sum is 57 parts of 60, which is the whole. |
| − | + | |style="text-align:right;"|ובין כולם הם נ"ז חלקים מס' שהוא השלם | |
| − | + | |- | |
| + | | colspan="2"| | ||
| + | :<math>\scriptstyle{\color{blue}{\frac{\left(\frac{1}{3}\sdot60\right)+\left(\frac{1}{4}\sdot60\right)+\left(\frac{1}{5}\sdot60\right)+\left(\frac{1}{6}\sdot60\right)}{60}=\frac{20+15+12+10}{60}=\frac{57}{60}}}</math> | ||
|- | |- | ||
| | | | ||
| − | + | :The 3 parts the remain from 57 to 60 are the body that weighs 10 liṭra. | |
| − | + | :<math>\scriptstyle{\color{blue}{\frac{60}{60}-\frac{57}{60}=\frac{3}{60}}}</math> | |
|style="text-align:right;"|ואותם הג' חלקים שנשארו מנ"ז עד ס' הוא הגוף ששוקל י' ליט‫' | |style="text-align:right;"|ואותם הג' חלקים שנשארו מנ"ז עד ס' הוא הגוף ששוקל י' ליט‫' | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> So, say: if 3 parts of 60, i.e. the body, weighs 10 liṭra, how much do the 57 parts of 60, which are the head and the tail weigh? | |
| + | :<math>\scriptstyle{\color{blue}{\frac{3}{60}:10=\frac{57}{60}:a}}</math> | ||
|style="text-align:right;"|אמו' א"כ אם ג' חלקים מס' דהיינו הגוף שוקל י' ליט' הנ"ז חלקים מס' שהם הראש והזנב כמה שוקל | |style="text-align:right;"|אמו' א"כ אם ג' חלקים מס' דהיינו הגוף שוקל י' ליט' הנ"ז חלקים מס' שהם הראש והזנב כמה שוקל | ||
|- | |- | ||
| | | | ||
| − | ::the | + | :Say: 57 times 10 is 570. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אמו' נ"ז פעמ' י' הם תק"ע |
| − | + | |- | |
| + | | | ||
| + | :Divide it by 3; the result is 190. | ||
| + | :<math>\scriptstyle{\color{blue}{a=\frac{57\sdot10}{3}=\frac{570}{3}=190}}</math> | ||
| + | |style="text-align:right;"|חלקם בג' יבואו ק"צ | ||
| + | |- | ||
| + | | | ||
| + | :We find that the head and the tail weigh 190 liṭra and the body weighs 10 liṭra. | ||
| + | |style="text-align:right;"|נמצא שהראש והזנב שוקלים ק"צ ליט' והגוף שוקל י' ליט‫' | ||
| + | |- | ||
| + | | | ||
| + | :Hence, the whole fish weighs two hundred liṭra. | ||
| + | :<math>\scriptstyle{\color{blue}{x=190+10=200}}</math> | ||
| + | |style="text-align:right;"|הרי שכל הדג שקל מאתים ליט‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | :If you wish to know how much does the head weigh: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואם תרצה לדעת כמה שוקל הראש |
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :You already know that the head is a third and a quarter of the whole fish, which are 20 and 15 parts of 60 and their sum is 35. |
| − | + | :<math>\scriptstyle{\color{blue}{\frac{\left(\frac{1}{3}\sdot60\right)+\left(\frac{1}{4}\sdot60\right)}{60}=\frac{20+15}{60}=\frac{35}{60}}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כבר ידעת שהראש השליש והרביע מכל הדג שהם כ' וט"ו חלקים מס' ובין כולם ל"ה |
| − | כבר ידעת שהראש השליש והרביע מכל הדג שהם כ' וט"ו חלקים מס' ובין כולם ל"ה | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> Say: if 3 parts of 60, which are the body, weigh 10 liṭra, how much do the 35 parts of 60 [weigh]? | |
| + | :<math>\scriptstyle{\color{blue}{\frac{3}{60}:10=\frac{35}{60}:b}}</math> | ||
|style="text-align:right;"|ואמו' אם ג' חלקים מס' שהוא הגוף שוקל י' ליט' ל"ה חלקים מס' כמה הם | |style="text-align:right;"|ואמו' אם ג' חלקים מס' שהוא הגוף שוקל י' ליט' ל"ה חלקים מס' כמה הם | ||
|- | |- | ||
| | | | ||
| − | ::the head | + | :Say: 10 times 35 is 350. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אמו' י' פעמ' ל"ה ש"נ |
| − | חלקם בג' יבואו קי"ו ליט' וב' שלישייות והוא משק' הראש | + | |- |
| + | | | ||
| + | :Divide it by 3; the result is 116 liṭra and 2-thirds, and this is the weight of the head. | ||
| + | :<math>\scriptstyle{\color{blue}{b=\frac{35\sdot10}{3}=\frac{350}{3}=116+\frac{2}{3}}}</math> | ||
| + | |style="text-align:right;"|חלקם בג' יבואו קי"ו ליט' וב' שלישייות והוא משק' הראש | ||
| + | |- | ||
| + | | | ||
| + | :If you wish to know how much does the tail weigh: | ||
| + | |style="text-align:right;"|ואם תרצה לדעת כמה שוקל הזנב | ||
|- | |- | ||
| | | | ||
| − | : | + | :You already know that the tail weighs one-fifth and one-sixth of 60, which are 12 and 10 and their sum is 22 parts of 60. |
| − | + | :<math>\scriptstyle{\color{blue}{\frac{\left(\frac{1}{5}\sdot60\right)+\left(\frac{1}{6}\sdot60\right)}{60}=\frac{12+10}{60}=\frac{22}{60}}}</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כבר ידעת שהזנב שוקל החומש והשתות מס' שהם י"ב וי' ובין כולם הוא כ"ב חלקים מס‫' |
| − | כבר ידעת שהזנב שוקל החומש והשתות מס' שהם י"ב וי' ובין כולם הוא כ"ב חלקים מס‫' | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Rule of Three:'''</span> Say: if 3 parts of 60 weigh 10 liṭra, how much do the 22 parts of 60 weigh? | |
| + | :<math>\scriptstyle{\color{blue}{\frac{3}{60}:10=\frac{22}{60}:c}}</math> | ||
|style="text-align:right;"|ואמו' אם ג' חלקים מס' שוקל י' ליט' כ"ב חלקים מס' כמה שוקלים | |style="text-align:right;"|ואמו' אם ג' חלקים מס' שוקל י' ליט' כ"ב חלקים מס' כמה שוקלים | ||
|- | |- | ||
| | | | ||
| − | :: | + | :Say: 22 times 10 is 220. |
| − | |style="text-align:right;"|אמו' כ"ב פעמ' י' הם ר"כ | + | |style="text-align:right;"|אמו' כ"ב פעמ' י' הם ר"כ |
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :Divide it by 3; the result is 73 liṭra and a third, and this is the weight of the tail. |
| − | + | :<math>\scriptstyle{\color{blue}{c=\frac{22\sdot10}{3}=\frac{220}{3}=73+\frac{1}{3}}}</math> | |
| − | |style="text-align:right;"|נמצא שהגוף שוקל י' ליט' והראש שוקל קי"ו ליט' וב' שלשייו' והזנב שוקל ע"ג ליט' ושליש< | + | |style="text-align:right;"|חלקם בג' יבואו ע"ג ליט' ושליש והוא משקל הזנב |
| − | סך הכל מאתים ליט‫' | + | |- |
| + | | | ||
| + | :We find that the body weighs 10 liṭra, the head weighs 116 liṭra and 2-thirds, and the tail weighs 73 liṭra and a third. | ||
| + | |style="text-align:right;"|נמצא שהגוף שוקל י' ליט' והראש שוקל קי"ו ליט' וב' שלשייו' והזנב שוקל ע"ג ליט' ושליש | ||
| + | |- | ||
| + | | | ||
| + | :The total is two hundred liṭra. | ||
| + | :<math>\scriptstyle{\color{blue}{10+\left(116+\frac{2}{3}\right)+\left(73+\frac{1}{3}\right)=200}}</math> | ||
| + | |style="text-align:right;"|סך הכל מאתים ליט‫' | ||
|- | |- | ||
| | | | ||
| − | === Divide a Quantity Problem - Proportional Division === | + | === <span style=color:green>Divide a Quantity Problem - Proportional Division</span> === |
| | | | ||
| Line 5,376: | Line 6,928: | ||
:You want to know: how much was the share of each of the fish and how much money did each of them have<br> | :You want to know: how much was the share of each of the fish and how much money did each of them have<br> | ||
:<math>\scriptstyle\frac{1}{2}X+\frac{1}{3}X+\frac{1}{9}X=9</math> | :<math>\scriptstyle\frac{1}{2}X+\frac{1}{3}X+\frac{1}{9}X=9</math> | ||
| − | |style="text-align:right;"|הרי שג' אנשים קנו א' דג בט' פשוטי‫'<br> | + | |style="text-align:right;"|‫<ref>MS Ithaca om.</ref>הרי שג' אנשים קנו א' דג בט' פשוטי‫'<br> |
האחד יש לו החצי והשני יש לו השליש והשלישי יש לו התשיע<br> | האחד יש לו החצי והשני יש לו השליש והשלישי יש לו התשיע<br> | ||
ותרצה לידע כמה חלק מן הדג יש לכל אחד וכמה מעות יגיע לכל אחד{{#annotend:TBmC}} | ותרצה לידע כמה חלק מן הדג יש לכל אחד וכמה מעות יגיע לכל אחד{{#annotend:TBmC}} | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''False Position:'''</span> Do this way, say: a half, a third and a ninth are found in 18. | |
|style="text-align:right;"|עשה על זה הדרך אמור חצי ושליש ותשיע ימצאו בי"ח | |style="text-align:right;"|עשה על זה הדרך אמור חצי ושליש ותשיע ימצאו בי"ח | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Denominator:'''</span> Its half is 9; its third is 6; its ninth is 2. Sum them together; they are 17 and this is the denominator. | |
| + | :<math>\scriptstyle{\color{blue}{\left(\frac{1}{2}\sdot18\right)+\left(\frac{1}{3}\sdot18\right)+\left(\frac{1}{9}\sdot18\right)=9+6+2=17}}</math> | ||
|style="text-align:right;"|חציו ט' שלישיתו ו' תשיעיתו ב' חבר אותם יחד יהיו י"ז והוא המחלק | |style="text-align:right;"|חציו ט' שלישיתו ו' תשיעיתו ב' חבר אותם יחד יהיו י"ז והוא המחלק | ||
|- | |- | ||
| | | | ||
| − | : | + | :For the one who has a half of the fish, say: the half of 18 is 9. Divide it by 17: his share of the fish is 9 parts of 17. |
| + | :<math>\scriptstyle{\color{blue}{\frac{1\sdot\left(\frac{1}{2}\sdot18\right)}{17}=\frac{9}{17}}}</math> | ||
|style="text-align:right;"|ואותו שיש לו החצי מן הדג<br> | |style="text-align:right;"|ואותו שיש לו החצי מן הדג<br> | ||
אמו' החצי מי"ח הוא ט‫'<br> | אמו' החצי מי"ח הוא ט‫'<br> | ||
| Line 5,395: | Line 6,949: | ||
|- | |- | ||
| | | | ||
| − | : | + | :If you want to know how much he paid for his share, which is the half: |
| − | |style="text-align:right;"|ואם תרצה לידע כמה ופרע בעבור חלקו שהוא החצי | + | |style="text-align:right;"|ואם תרצה לידע כמה ופרע בעבור חלקו שהוא החצי |
| − | אמו' החצי מי"ח הוא ט' ותרבה אותו בט' שהוא סך מכירת הדג יבא פ"א | + | |- |
| − | + | | | |
| + | :Say: a half of 18 is 9; multiply it by 9, which is the total price of the fish; the result is 81. | ||
| + | |style="text-align:right;"|אמו' החצי מי"ח הוא ט' ותרבה אותו בט' שהוא סך מכירת הדג יבא פ"א | ||
|- | |- | ||
| | | | ||
| − | ::the one who has a third: <math>\scriptstyle{\color{blue}{\frac{1\sdot\left(\frac{1}{3}\sdot18\right)}{17}=\frac{1\sdot6}{17}=\frac{6}{17}}}</math> | + | :Divide it by 17; the result is 4 pešuṭim and 13 parts of 17 of one pašuṭ. |
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{2}X=\frac{9\sdot9}{17}=\frac{81}{17}=4+\frac{13}{17}}}</math> | ||
| + | |style="text-align:right;"|חלקם בי"ז יבוא ד' פשוטי' וי"ג חלקים מי"ז מן הפשוט | ||
| + | |- | ||
| + | | | ||
| + | :For the one who has a third of the fish, say: the third of 18 is 6. Multiply it by the fish, which is 1; say: 6 times 1 is 6. Divide it by 17: his share of the fish is 6 parts of 17. | ||
| + | :<math>\scriptstyle{\color{blue}{\frac{1\sdot\left(\frac{1}{3}\sdot18\right)}{17}=\frac{1\sdot6}{17}=\frac{6}{17}}}</math> | ||
|style="text-align:right;"|ואותו שיש לו השליש מן הדג<br> | |style="text-align:right;"|ואותו שיש לו השליש מן הדג<br> | ||
אמו' השליש מי"ח הוא ו' ותרבה אותו על הדג שהוא א' ואמו' ו' פעמ' א' ו‫'<br> | אמו' השליש מי"ח הוא ו' ותרבה אותו על הדג שהוא א' ואמו' ו' פעמ' א' ו‫'<br> | ||
| Line 5,407: | Line 6,969: | ||
|- | |- | ||
| | | | ||
| − | : | + | :If you want to know how much money he paid for his share, which is the third: |
| − | |style="text-align:right;"|ואם תרצה לידע כמה מעות יפרע בעבור חלקו שהוא השליש | + | |style="text-align:right;"|ואם תרצה לידע כמה מעות יפרע בעבור חלקו שהוא השליש |
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | ::the one who has a ninth: <math>\scriptstyle{\color{blue}{\frac{1\sdot\left(\frac{1}{9}\sdot18\right)}{17}=\frac{1\sdot2}{17}=\frac{ | + | :Say: a third of 18 is 6; multiply it by 9 pešuṭim, which is the price of the fish; say: 6 times 9 is 54. |
| + | |style="text-align:right;"|אמור השליש מי"ח הוא ו' ותרבה אותם על ט' פשוטי' שנמכר הדג ואמו' ו' פעמ' ט' הם נ"ד | ||
| + | |- | ||
| + | | | ||
| + | :Divide it by 17: he paid for the third 3 pešuṭim and 3 parts of 17. | ||
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{3}X=\frac{6\sdot9}{17}=\frac{54}{17}=3+\frac{3}{17}}}</math> | ||
| + | |style="text-align:right;"|חלקם בי"ז הרי שיפרע בעבור השליש ג' פשוטי' וג' חלקים מי"ז | ||
| + | |- | ||
| + | | | ||
| + | :For the one who has a ninth [of the fish], say: the ninth of 1[8] is 2. Multiply it by 1, which is the fish; say: 2 times 1 is 2. Divide it by 17: his share of the fish is 2 parts of 17. | ||
| + | :<math>\scriptstyle{\color{blue}{\frac{1\sdot\left(\frac{1}{9}\sdot18\right)}{17}=\frac{1\sdot2}{17}=\frac{2}{17}}}</math> | ||
|style="text-align:right;"|ואותו שיש לו התשיע<br> | |style="text-align:right;"|ואותו שיש לו התשיע<br> | ||
אמו' התשיע מי"ז הוא ב' ותרבה אותם על א' שהוא הדג ואמו' ב' פעמ' א' ב‫'<br> | אמו' התשיע מי"ז הוא ב' ותרבה אותם על א' שהוא הדג ואמו' ב' פעמ' א' ב‫'<br> | ||
| Line 5,419: | Line 6,989: | ||
|- | |- | ||
| | | | ||
| − | : | + | :If you want to know how much money he paid for his share, which is the ninth: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואם תרצה לידע כמה מעות יפרע בעבו' חלקו שהוא התשיע |
| − | + | |- | |
| − | חלקם בי"ז יבא א' פשוט' וא' חלק מי"ז | + | | |
| − | + | :Say: a ninth of 18 is 2; multiply it by the price of the fish, which is 9; say: 2 times 9 is 18. | |
| − | + | |style="text-align:right;"|אמו' התשיע מי"ח הוא ב' ותרבה אותם על מה שנמכר הדג שהוא ט' ואמו' ב' פעמ' ט' הם י"ח | |
| + | |- | ||
| + | | | ||
| + | :Divide it by 17; the result is 1 pašuṭ and 1 part of 17. | ||
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{9}X=\frac{2\sdot9}{17}=\frac{18}{17}=1+\frac{1}{17}}}</math> | ||
| + | |style="text-align:right;"|חלקם בי"ז יבא א' פשוט' וא' חלק מי"ז | ||
|- | |- | ||
| | | | ||
| − | === Buy and Sell Problem === | + | === <span style=color:green>Buy and Sell Problem</span> === |
| | | | ||
| Line 5,435: | Line 7,010: | ||
*{{#annot:figs|641|JfiA}}A man said to his friend: here are two baskets of figs, each contains 100 figs. | *{{#annot:figs|641|JfiA}}A man said to his friend: here are two baskets of figs, each contains 100 figs. | ||
:Sell the fine fruit at 20 for one pašuṭ, and the defective fruit at 30 for one pašuṭ. | :Sell the fine fruit at 20 for one pašuṭ, and the defective fruit at 30 for one pašuṭ. | ||
| − | :Their total was | + | :Their total was 8 pešuṭim and a third. |
:One asked him: how do you sell [the figs]? | :One asked him: how do you sell [the figs]? | ||
:He answered him: these at 30 for one pašuṭ and these at 20 for one pašuṭ. | :He answered him: these at 30 for one pašuṭ and these at 20 for one pašuṭ. | ||
:He replied: if so, give me from these and from these at 50 for 2 pešuṭim. | :He replied: if so, give me from these and from these at 50 for 2 pešuṭim. | ||
| − | :He sold all for 8 pešuṭim and lost | + | :He sold all for 8 pešuṭim and lost a third of a pašuṭ. |
:<math>\scriptstyle{\color{blue}{\left(\frac{100}{20}+\frac{100}{30}\right)-8=\left[5+\left(3+\frac{1}{3}\right)\right]-8=\left(8+\frac{1}{3}\right)-8=\frac{1}{3}}}</math> | :<math>\scriptstyle{\color{blue}{\left(\frac{100}{20}+\frac{100}{30}\right)-8=\left[5+\left(3+\frac{1}{3}\right)\right]-8=\left(8+\frac{1}{3}\right)-8=\frac{1}{3}}}</math> | ||
| − | |style="text-align:right;"|אדם אחד אמר לחבירו הנה שני כלכלות של תאנים בכל אחת מהן ק' תאנים<br> | + | |style="text-align:right;"|<big>אדם אחד אמר לחבירו הנה שני</big> כלכלות של תאנים בכל אחת מהן ק' תאנים<br> |
מכור היפות כ' בפשוט והרעות ל' בפשוט עולה חשבונם ח' פשיטי ושליש<br> | מכור היפות כ' בפשוט והרעות ל' בפשוט עולה חשבונם ח' פשיטי ושליש<br> | ||
אמר לו אחד היאך אתה מוכר<br> | אמר לו אחד היאך אתה מוכר<br> | ||
| Line 5,449: | Line 7,024: | ||
|- | |- | ||
| | | | ||
| − | : | + | :Because, those [whose price is] 20 for one pašuṭ cost 5 pešiṭim. |
| + | :<math>\scriptstyle{\color{blue}{\frac{100}{20}=5}}</math> | ||
|style="text-align:right;"|כי אותם שהם כ' בפשוט עולי' ה' פשיטים | |style="text-align:right;"|כי אותם שהם כ' בפשוט עולי' ה' פשיטים | ||
|- | |- | ||
| | | | ||
| − | : | + | :And those [whose price is] 30 for one pašuṭ cost 3 [pešiṭim] and a third of a pašuṭ. |
| + | :<math>\scriptstyle{\color{blue}{\frac{100}{30}=3+\frac{1}{3}}}</math> | ||
|style="text-align:right;"|ואותם שהם ל' בפשוט עולי' ג' פעמי' ושליש פשוט | |style="text-align:right;"|ואותם שהם ל' בפשוט עולי' ג' פעמי' ושליש פשוט | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | === Ordering Problems === | + | === <span style=color:green>Ordering Problems</span> === |
| | | | ||
| Line 5,470: | Line 7,045: | ||
:How much should [each of] these coins [be worth]? | :How much should [each of] these coins [be worth]? | ||
:<math>\scriptstyle{\color{blue}{1+2+3+4+8+12=30}}</math> | :<math>\scriptstyle{\color{blue}{1+2+3+4+8+12=30}}</math> | ||
| − | |style="text-align:right;"|אדם שיש לו שכיר אחד לשלשים יום בל' פשיטי' ורוצה לקבל פרעונו בכל יום<br> | + | |style="text-align:right;"|<big>אדם שיש לו שכיר אחד לשלשים יום</big> בל' פשיטי' ורוצה לקבל פרעונו בכל יום<br> |
ואי' לשוכר רק ו' מטבעי' שוי' ל' פשיטי' בין כלם<br> | ואי' לשוכר רק ו' מטבעי' שוי' ל' פשיטי' בין כלם<br> | ||
| − | והוא פורע אותו בכל יום עם אלו המטבעו' הו' מטבעות ואינו מחסר | + | והוא פורע אותו בכל יום עם אלו המטבעו' הו' מטבעות ‫<ref>45r</ref>ואינו מחסר ואינו מותיר לו על מה שיש לו לקבל<br> |
היאך יהיו אלו המטבעות{{#annotend:Wv90}} | היאך יהיו אלו המטבעות{{#annotend:Wv90}} | ||
|- | |- | ||
| | | | ||
| + | :They should be according to this order: | ||
|style="text-align:right;"|צריכין להיות על זה הסדר | |style="text-align:right;"|צריכין להיות על זה הסדר | ||
|- | |- | ||
| | | | ||
| − | : | + | :Put 1 for the first; 2 for the second; 3 for the third; 4 for the fourth; 8 for the fifth; and 12 for the sixth. |
| − | |style="text-align:right;"|שהאחת שי[ם] א | + | |style="text-align:right;"|שהאחת שי[ם] א' והשנית ב' והשלישית ג' והרביעית ד' והחמשית ח' והששית י"ב |
|- | |- | ||
| | | | ||
| − | : | + | :By this the matter can be resolved. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ובזה יתכן העניין |
|- | |- | ||
| | | | ||
| − | : | + | |
| − | + | === <span style=color:green><span style=color:green>Multiple Quantities Problems</span></span> === | |
| − | + | ||
| | | | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | + | ==== <span style=color:green>Four Coins</span> ==== | |
| − | === | ||
| | | | ||
| Line 5,518: | Line 7,080: | ||
:The fourth is equal to a fifth of the third. | :The fourth is equal to a fifth of the third. | ||
:How much does each [of the coins] worth? | :How much does each [of the coins] worth? | ||
| − | |style="text-align:right;"|ד' מטבעות שוים בין ארבעתן ק' פשוטי‫'<br> | + | |style="text-align:right;"|‫<ref>MS Ithaca om.</ref>ד' מטבעות שוים בין ארבעתן ק' פשוטי‫'<br> |
הראשון שוה שליש ורביע ושתות מן השני<br> | הראשון שוה שליש ורביע ושתות מן השני<br> | ||
מה שנשאר מן השני שוה ב' חמישייו' מן השלישי<br> | מה שנשאר מן השני שוה ב' חמישייו' מן השלישי<br> | ||
| Line 5,524: | Line 7,086: | ||
הרביעי שוה חומש השלישי<br> | הרביעי שוה חומש השלישי<br> | ||
כמה שוה כל אחד{{#annotend:I5ZX}} | כמה שוה כל אחד{{#annotend:I5ZX}} | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | :<math>\scriptstyle\begin{cases}\scriptstyle a_1+a_2+a_3+a_4=100\\\scriptstyle a_1=\left(\frac{1}{3}+\frac{1}{4}+\frac{1}{6}\right)\sdot a_2\\\scriptstyle a_2-\left[\left(\frac{1}{3}+\frac{1}{4}+\frac{1}{6}\right)\sdot a_2\right]=\frac{2}{5}a_3\\\scriptstyle a_3=\left(\frac{1}{2}+\frac{1}{3}\right)\sdot a_1\\\scriptstyle a_4=\frac{1}{5}a_3\end{cases}</math> | ||
|- | |- | ||
| | | | ||
| − | : | + | :For the first that is equal to a third, a quarter, and a sixth of the second, say: how many parts of the whole are they? |
| + | |style="text-align:right;"|אמו' הראשון ששוה שליש ורביע ושתות מן השני כמה חלקים משלם הם | ||
| + | |- | ||
| | | | ||
| + | :Say: a third, a quarter, and a sixth are found in 12. | ||
| + | |style="text-align:right;"|אמו' שליש ורביע ושתות ימצאו בי"ב | ||
|- | |- | ||
| | | | ||
| − | :::<math>\scriptstyle{\color{blue}{ | + | :The third is 4; the quarter is 3; the sixth is 2. Their total is 9 parts of 12, which is 3-quarters. |
| − | + | |style="text-align:right;"|השליש ד' הרביע ג' השתות ב' והם בן כולם ט' חלקים מי"ב שהם ג' רביעייו‫' | |
| − | + | |- | |
| − | + | | colspan="2"| | |
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{3}+\frac{1}{4}+\frac{1}{6}=\frac{\left(\frac{1}{3}\sdot12\right)+\left(\frac{1}{4}\sdot12\right)+\left(\frac{1}{6}\sdot12\right)}{12}=\frac{4+3+2}{12}=\frac{9}{12}=\frac{3}{4}}}</math> | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{a_1=\left(\frac{1}{3}+\frac{1}{4}+\frac{1}{6}\right)\sdot a_2=\frac{3}{4}a_2}}</math> | + | :Therefore, the first is equal to 3-quarters of the second. |
| + | :<math>\scriptstyle{\color{blue}{a_1=\left(\frac{1}{3}+\frac{1}{4}+\frac{1}{6}\right)\sdot a_2=\frac{3}{4}a_2}}</math> | ||
|style="text-align:right;"|אם כן הראשון שוה ג' רביעייו' מהשני | |style="text-align:right;"|אם כן הראשון שוה ג' רביעייו' מהשני | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\begin{cases}\scriptstyle a_2=40\\\scriptstyle a_1=\frac{3}{4}a_2=\frac{3}{4}\sdot40=30\\\scriptstyle a_3=\left(\frac{1}{2}+\frac{1}{3}\right)a_1=\left(\frac{1}{2}+\frac{1}{3}\right)\sdot30=25\\\scriptstyle a_4=\frac{1}{5}a_3=\frac{1}{5}\sdot25=5\\\scriptstyle a_1+a_2+a_3+a_4=30+40+25+5=100\end{cases}}}</math> | + | :All these fractions are found in forty: |
| − | | | + | |style="text-align:right;"|וימצאו כל אילו השברים בארבעים |
| − | + | |- | |
| − | + | | | |
| − | + | :So, the second is 40. | |
| − | + | |style="text-align:right;"|אם כן השני הוא מ‫' | |
| − | + | |- | |
| + | | | ||
| + | :The first, which is equal to 3-quarters of the second, is 30. | ||
| + | |style="text-align:right;"|הראשון ששווה ג' רביעייו' מהשני הוא ל‫' | ||
| + | |- | ||
| + | | | ||
| + | :The third that is equal to a half and a third of the first, which is 30, is 25. | ||
| + | |style="text-align:right;"|השלישי ששוה חצי ושליש מן הראשון שהוא ל' הוא כ"ה | ||
| + | |- | ||
| + | | | ||
| + | :The fourth that is equal to a fifth of the third, which is 25, is 5. | ||
| + | |style="text-align:right;"|הרביעי ששוה חומש השלישי שהוא כ"ה הוא ה‫' | ||
| + | |- | ||
| + | | | ||
| + | :If you sum up all these numbers, they are 100. | ||
| + | |style="text-align:right;"|ואם תקבץ כל אלו המספרים יהיו ק‫' | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | :<math>\scriptstyle{\color{blue}{\begin{cases}\scriptstyle a_2=40\\\scriptstyle a_1=\frac{3}{4}a_2=\frac{3}{4}\sdot40=30\\\scriptstyle a_3=\left(\frac{1}{2}+\frac{1}{3}\right)a_1=\left(\frac{1}{2}+\frac{1}{3}\right)\sdot30=25\\\scriptstyle a_4=\frac{1}{5}a_3=\frac{1}{5}\sdot25=5\\\scriptstyle a_1+a_2+a_3+a_4=30+40+25+5=100\end{cases}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | |||
| + | ==== <span style=color:green>Selling Eggs</span> ==== | ||
| + | |||
| + | | | ||
|- | |- | ||
| | | | ||
| Line 5,552: | Line 7,147: | ||
:He said to them: go, sell the eggs equally, and bring me equal amounts of money. | :He said to them: go, sell the eggs equally, and bring me equal amounts of money. | ||
:<math>\scriptstyle10X=30Y=50Z</math> | :<math>\scriptstyle10X=30Y=50Z</math> | ||
| − | |style="text-align:right;"|אדם אחד נתן לשלשת בניו ביצות למכור<br> | + | |style="text-align:right;"|<big>אדם אחד נתן לשלשת בניו ביצות למכור</big><br> |
לאחד נתן נ' ביצות ולשלישי ל' ולשלישי י‫'<br> | לאחד נתן נ' ביצות ולשלישי ל' ולשלישי י‫'<br> | ||
ואמר להם לכו ומכרו הביצות בשוה והביאו לי מעות בשוה{{#annotend:hBdI}} | ואמר להם לכו ומכרו הביצות בשוה והביאו לי מעות בשוה{{#annotend:hBdI}} | ||
|- | |- | ||
| | | | ||
| − | : | + | :[First], they sold 7 eggs for one pašuṭ: |
|style="text-align:right;"|הלכו ונתנו ז' ביצות בפשוט | |style="text-align:right;"|הלכו ונתנו ז' ביצות בפשוט | ||
|- | |- | ||
| | | | ||
| − | : | + | :The one who had 50 eggs sold 49 eggs for 7 pešuṭim and had one egg left. |
| − | + | :<math>\scriptstyle{\color{blue}{50-\left(7\sdot7\right)=50-49=1}}</math> | |
|style="text-align:right;"|אותו שהיו לו נ' ביצות מכר ז' פ' מ"ט ביצות בז' פשיטי' ונשארה לו ביצה אחת | |style="text-align:right;"|אותו שהיו לו נ' ביצות מכר ז' פ' מ"ט ביצות בז' פשיטי' ונשארה לו ביצה אחת | ||
|- | |- | ||
| | | | ||
| − | : | + | :The one who had 30 eggs sold 28 eggs for 4 pešuṭim and had 2 eggs left. |
| − | + | :<math>\scriptstyle{\color{blue}{30-\left(7\sdot4\right)=30-28=2}}</math> | |
|style="text-align:right;"|ואותו שהיו לו ל' ביצות מכר כ"ח ביצות בד"פ ונשארו לו ב' ביצות | |style="text-align:right;"|ואותו שהיו לו ל' ביצות מכר כ"ח ביצות בד"פ ונשארו לו ב' ביצות | ||
|- | |- | ||
| | | | ||
| − | : | + | :The one who had 10 eggs sold [7] eggs for [one] pašuṭ and had 3 eggs left. |
| − | + | :<math>\scriptstyle{\color{blue}{10-\left(7\sdot1\right)=10-7=3}}</math> | |
|style="text-align:right;"|ואותו שהיו לו י' ביצות מכר א' ביצה בז' פשיטי' ונשארו לו ג' ביצי‫' | |style="text-align:right;"|ואותו שהיו לו י' ביצות מכר א' ביצה בז' פשיטי' ונשארו לו ג' ביצי‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | :Then, they sold the eggs they had left at 3 pešuṭim for an egg: |
|style="text-align:right;"|הלכו פעם שניה ומכרו הביצות שנשארו בג' פשיטי' הביצה | |style="text-align:right;"|הלכו פעם שניה ומכרו הביצות שנשארו בג' פשיטי' הביצה | ||
|- | |- | ||
| | | | ||
| − | : | + | :The first who had one egg left received 3 pešuṭim; with the 7 pešuṭim he received from the first sale, he received 10 pešuṭim. |
| − | : | + | :<math>\scriptstyle{\color{blue}{\left(1\sdot3\right)+7=3+7=10}}</math> |
| − | |style="text-align:right;"|הראשון שנשאר לו ביצה אחת קיבל ג"פ וז"פ שקיבל במכירה הראשונה<br> | + | |style="text-align:right;"|הראשון <s>ה</s> שנשאר לו ביצה אחת קיבל ג"פ וז"פ שקיבל במכירה הראשונה<br> |
הרי שקיבל י' פשיטי‫' | הרי שקיבל י' פשיטי‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | :The second who had 2 eggs left received 6 pešuṭim; with the 4 pešuṭim of the first sale, he received 10 pešuṭim. |
| − | : | + | :<math>\scriptstyle{\color{blue}{\left(2\sdot3\right)+4=6+4=10}}</math> |
|style="text-align:right;"|והשיני שנשארו לו ב' ביצות קיבל ו' פשיטי' וד"פ שקיבל במכירה ראשונה<br> | |style="text-align:right;"|והשיני שנשארו לו ב' ביצות קיבל ו' פשיטי' וד"פ שקיבל במכירה ראשונה<br> | ||
הרי שקבל י' פשיטי‫' | הרי שקבל י' פשיטי‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | :The third who had 3 eggs left received 9 pešuṭim; with the 1 pešuṭ he received from the first sale he got 10 pešuṭim. |
| − | : | + | :<math>\scriptstyle{\color{blue}{\left(3\sdot3\right)+1=9+1=10}}</math> |
| − | |style="text-align:right;"|והשלישי שנשארו ג' ביצות קיבל ט' פשיטי' וא' פשוט שקיבל במכירה הראשונה<br> | + | |style="text-align:right;"|והשלישי שנשארו ג' <s>פשיטי'</s> ביצות קיבל ט' פשיטי' וא' פשוט שקיבל במכירה הראשונה<br> |
הרי שקיבל י"פ | הרי שקיבל י"פ | ||
|- | |- | ||
| | | | ||
| − | : | + | :So, they sold the eggs for the same price and received the same amount of money. |
| − | |style="text-align:right;"|הרי שכלם מכרו הביצות בשוה וכלם קבלו המעות בשוה | + | |style="text-align:right;"|הרי <s>שקיבל</s> שכלם מכרו הביצות בשוה וכלם קבלו המעות בשוה |
| + | |- | ||
| + | | | ||
| + | ==== <span style=color:green>Four Coins</span> ==== | ||
| + | |||
| + | | | ||
|- | |- | ||
| | | | ||
*{{#annot:four coins|652|vSHO}}Four coins are worth all in all 80 pešuṭim: | *{{#annot:four coins|652|vSHO}}Four coins are worth all in all 80 pešuṭim: | ||
:The first is equal to a half and a sixth of the second. | :The first is equal to a half and a sixth of the second. | ||
| − | :What is left from the second equals | + | :What is left from the second equals 2-thirds of the third. |
:The third is equal to a third, a quarter, and a sixth of the first. | :The third is equal to a third, a quarter, and a sixth of the first. | ||
| − | :The fourth is equal to | + | :The fourth is equal to 4-fifths of the [third]. |
:How much does each [of the coins] worth? | :How much does each [of the coins] worth? | ||
| − | |style="text-align:right;"|ד' מט' מטבעות שוים בין ארבעתם פ' פשוטי‫'<br> | + | |style="text-align:right;"|‫<ref>MS Ithaca om.</ref>ד' מט' מטבעות שוים בין ארבעתם פ' פשוטי‫'<br> |
הראשון שוה החצי והשתות מן השני<br> | הראשון שוה החצי והשתות מן השני<br> | ||
הנשאר מן השני הם ב' שלישיות מן השלישי<br> | הנשאר מן השני הם ב' שלישיות מן השלישי<br> | ||
השלישי הוא שליש ורביע ושתות מן הראשו‫'<br> | השלישי הוא שליש ורביע ושתות מן הראשו‫'<br> | ||
| − | הרביעי הוא ד' חומשים | + | הרביעי הוא ד' חומשים מהשני<br> |
ותרצה לידע כמה שוה כל אחד ואחד{{#annotend:vSHO}} | ותרצה לידע כמה שוה כל אחד ואחד{{#annotend:vSHO}} | ||
|- | |- | ||
| − | | | + | | colspan="2"| |
:<math>\scriptstyle\begin{cases}\scriptstyle a_1+a_2+a_3+a_4=80\\\scriptstyle a_1=\left(\frac{1}{2}+\frac{1}{6}\right)\sdot a_2\\\scriptstyle a_2-\left[\left(\frac{1}{2}+\frac{1}{6}\right)\sdot a_2\right]=\frac{2}{3}a_3\\\scriptstyle a_3=\left(\frac{1}{3}+\frac{1}{4}+\frac{1}{6}\right)\sdot a_1\\\scriptstyle a_4=\frac{4}{5}a_3\end{cases}</math> | :<math>\scriptstyle\begin{cases}\scriptstyle a_1+a_2+a_3+a_4=80\\\scriptstyle a_1=\left(\frac{1}{2}+\frac{1}{6}\right)\sdot a_2\\\scriptstyle a_2-\left[\left(\frac{1}{2}+\frac{1}{6}\right)\sdot a_2\right]=\frac{2}{3}a_3\\\scriptstyle a_3=\left(\frac{1}{3}+\frac{1}{4}+\frac{1}{6}\right)\sdot a_1\\\scriptstyle a_4=\frac{4}{5}a_3\end{cases}</math> | ||
| + | |- | ||
| | | | ||
| + | :Do it this way: | ||
| + | |style="text-align:right;"|עשה על זה הדרך | ||
|- | |- | ||
| | | | ||
| − | :: | + | :Since the first is equal to a half and a sixth of the second, say: a half and a sixth are found in 6. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|בעבו' שהראשו' שוה החצי והשתות מהשני<br> |
| − | אמור חצי ושתות ימצאו בו‫' | + | אמור חצי ושתות ימצאו בו‫' |
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{a_1=\left(\frac{1}{2}+\frac{1}{6}\right)\sdot a_2=\frac{2}{3}a_2}}</math> | + | :The half is 3; the sixth is 1; their sum is 4; so they are 2-thirds. |
| + | |style="text-align:right;"|החצי ג' השתות א' וכללם ד' והם ב' שלשיות | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | :<math>\scriptstyle{\color{blue}{\frac{1}{2}+\frac{1}{6}=\frac{\left(\frac{1}{2}\sdot6\right)+\left(\frac{1}{6}\sdot6\right)}{6}=\frac{3+1}{6}=\frac{4}{6}=\frac{2}{3}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :Therefore, the one that is equal to a half and a sixth of the second is as saying 2-thirds of the second. | ||
| + | :<math>\scriptstyle{\color{blue}{a_1=\left(\frac{1}{2}+\frac{1}{6}\right)\sdot a_2=\frac{2}{3}a_2}}</math> | ||
|style="text-align:right;"|אם כן זה ששוה חצי ושתות מן השני הוא כאלו אמר שוה ב' שלישייו' מן השני | |style="text-align:right;"|אם כן זה ששוה חצי ושתות מן השני הוא כאלו אמר שוה ב' שלישייו' מן השני | ||
|- | |- | ||
| | | | ||
| − | :: | + | :The third is equal to a third, a quarter, and a sixth of the first. Say: a third, a quarter, and a sixth are found in 60. |
|style="text-align:right;"|והשלישי ששוה שליש ורביע ושתות מן הראשו‫'<br> | |style="text-align:right;"|והשלישי ששוה שליש ורביע ושתות מן הראשו‫'<br> | ||
| − | אמו' שליש ורביע ושתות ימצאו בס‫' | + | אמו' שליש ורביע ושתות ימצאו בס‫' |
| − | השליש כ' הרביע ט"ו השתות י | + | |- |
| − | וכללם מ"ה חלקים מס' שהם ג' רביעייו' מס‫' | + | | |
| + | :The third is 20; the quarter is 15; the sixth is 10; their sum is 45 parts of 60, which are 3-quarters. | ||
| + | |style="text-align:right;"|השליש כ' הרביע ט"ו השתות י' וכללם מ"ה חלקים מס' שהם ג' רביעייו' מס‫' | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | :<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle\frac{1}{3}+\frac{1}{4}+\frac{1}{6}&\scriptstyle=\frac{\left(\frac{1}{3}\sdot60\right)+\left(\frac{1}{4}\sdot60\right)+\left(\frac{1}{6}\sdot60\right)}{60}\\&\scriptstyle=\frac{20+15+10}{60}=\frac{45}{60}=\frac{3}{4}\\\end{align}}}</math> | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{a_3=\frac{3}{4}a_1}}</math> | + | :It is as saying: the third is equal to 3-quarters of the first. |
| + | :<math>\scriptstyle{\color{blue}{a_3=\frac{3}{4}a_1}}</math> | ||
|style="text-align:right;"|והוא כאלו אמר השלישי שוה ג' רבעייו' מן הראשון | |style="text-align:right;"|והוא כאלו אמר השלישי שוה ג' רבעייו' מן הראשון | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{a_4=\frac{4}{5}a_3}}</math> | + | :The fourth is equal to 4-fifths of the third; this is clear. |
| + | :<math>\scriptstyle{\color{blue}{a_4=\frac{4}{5}a_3}}</math> | ||
|style="text-align:right;"|והרביעי שוה ד' חמישייו' מהשלישי הוא מבואר | |style="text-align:right;"|והרביעי שוה ד' חמישייו' מהשלישי הוא מבואר | ||
|- | |- | ||
| | | | ||
| − | :: | + | :So the order is as follows: |
| − | |style="text-align:right;"|אם כן הסדר הוא כך הראשו' שוה ב' שלשייו' מהשני | + | |style="text-align:right;"|אם כן הסדר הוא כך |
| − | והשלישי שוה ג' רביעייו' מן הראשון | + | |- |
| − | + | | | |
| + | :The first is equal to 2-thirds of the second. | ||
| + | |style="text-align:right;"|הראשו' שוה ב' שלשייו' מהשני | ||
| + | |- | ||
| + | | | ||
| + | :The third is equal to 3-quarters of the first. | ||
| + | |style="text-align:right;"|והשלישי שוה ג' רביעייו' מן הראשון | ||
|- | |- | ||
| | | | ||
| − | ::<span style=color: | + | :The fourth is equal to 4-fifths of the [third]. |
| + | |style="text-align:right;"|הרביעי שוה ד' חמישיו' מהשני | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | :<math>\scriptstyle{\color{blue}{\begin{cases}\scriptstyle a_1=\frac{2}{3}a_2\\\scriptstyle a_3=\frac{3}{4} a_1\\\scriptstyle a_4=\frac{4}{5}a_3\end{cases}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :<span style=color:green>'''False Position:'''</span> First, say: the fractions mentioned in this question, which are a half, a third, a quarter, and a sixth of a tenth, are found in 60. | ||
| + | :<math>\scriptstyle{\color{blue}{b_2=60}}</math> | ||
|style="text-align:right;"|ואמו' תחלה השברים הנזכרי' בזה החשבו' הם חצי ושליש ורביע ושתות מי' וימצאו כולם בס‫' | |style="text-align:right;"|ואמו' תחלה השברים הנזכרי' בזה החשבו' הם חצי ושליש ורביע ושתות מי' וימצאו כולם בס‫' | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\begin{cases}\scriptstyle b_2=60\\\scriptstyle b_1=\frac{2}{3}b_2=\frac{2}{3}\sdot60=40\\\scriptstyle b_3=\frac{3}{4}b_1=\frac{3}{4}\sdot40=30\\\scriptstyle b_4=\frac{4}{5}b_3=\frac{4}{5}\sdot30=24\end{cases}}}</math> | + | :Therefore, the second is 60. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אם כן השיני הוא ס‫' |
| − | + | |- | |
| − | + | | | |
| − | + | :The first, which is equal to 2-thirds of the second, is 40. | |
| + | |style="text-align:right;"|הראשון ששוה ב' שלישיו' מהשני הוא מ‫' | ||
| + | |- | ||
| + | | | ||
| + | :The third, which is equal to 3-quarters of the first, is 30. | ||
| + | |style="text-align:right;"|והשלישי ששוה ג' רביעיו' מהראשון הוא ל‫' | ||
| + | |- | ||
| + | | | ||
| + | :The fourth, which is equal to 4-fifths of the third, is 24. | ||
| + | |style="text-align:right;"|והרביעי ששוה ד' חמשייו' מהשלישי הוא כ"ד | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | :<math>\scriptstyle{\color{blue}{\begin{cases}\scriptstyle b_2=60\\\scriptstyle b_1=\frac{2}{3}b_2=\frac{2}{3}\sdot60=40\\\scriptstyle b_3=\frac{3}{4}b_1=\frac{3}{4}\sdot40=30\\\scriptstyle b_4=\frac{4}{5}b_3=\frac{4}{5}\sdot30=24\end{cases}}}</math> | ||
| + | |- | ||
| + | | | ||
| + | :The remainder from the second is 2-thirds of the third. | ||
| + | :<math>\scriptstyle{\color{blue}{b_2-b_1=60-40=20=\frac{2}{3}\sdot30=\frac{2}{3}b_3}}</math> | ||
| + | |style="text-align:right;"|והנשאר מן השני שהוא ב' שלישייו' מהשלישי | ||
| + | |- | ||
| + | | | ||
| + | :Proceed this way: | ||
| + | |style="text-align:right;"|עשה על זה הדרך | ||
| + | |- | ||
| + | | | ||
| + | :Say: how much is left from the second, which is 60, after you subtract from it the first, which is 40? 20 remains. | ||
| + | |style="text-align:right;"|אמו' כמה נשאר מהשני שהוא ס' אחר שהוצאת ממנו הראשון שהוא מ' נשארו כ‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | :Therefore, 20, which is remainder from the second, is 2-thirds of the third, which is 30. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אם כן כ' שהוא הנשאר מהשני הם ב' שלישייו' מהשלישי שהוא ל‫' |
| − | |||
| − | אם כן כ' שהוא הנשאר מהשני הם ב' שלישייו' מהשלישי שהוא ל‫' | ||
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''Denominator:'''</span> So, the first is 40, the second is 60, the third is 30, and the fourth is 24. Sum them up; they are 154 and this is the denominator. | |
| + | :<math>\scriptstyle{\color{blue}{b_1+b_2+b_3+b_4=40+60+30+24=154}}</math> | ||
|style="text-align:right;"|והרי שהראשו' הוא מ' והשני ס' והשלישי ל' והרביעי כ"ד<br> | |style="text-align:right;"|והרי שהראשו' הוא מ' והשני ס' והשלישי ל' והרביעי כ"ד<br> | ||
צרף אותם יחד יהיו קנ"ד והוא המחלק | צרף אותם יחד יהיו קנ"ד והוא המחלק | ||
|- | |- | ||
| | | | ||
| − | : | + | :<span style=color:green>'''Rule of Three:'''</span> If you wish to know how much the first is worth, say: 40 times 80 is 3 thousand and two hundred. |
| − | |style="text-align:right;"|ואם תרצה לדעת כמה שוה | + | |style="text-align:right;"|ואם תרצה לדעת כמה שוה הראשו' אמו' מ' פעמ' פ' ג' אלפים ומאתים |
| − | חלקם בקנ"ד יבואו | + | |- |
| + | | | ||
| + | :Divide it by 154; the result is 20 and 120 parts of 154. | ||
| + | :<math>\scriptstyle{\color{blue}{a_1=\frac{40\sdot80}{154}=\frac{3200}{154}=20+\frac{120}{154}}}</math> | ||
| + | |style="text-align:right;"|חלקם בקנ"ד יבואו כ' וק"כ חלקים מקנ"ד | ||
| + | |- | ||
| + | | | ||
| + | :<span style=color:green>'''Rule of Three:'''</span> If you wish to know how much the second is worth, say: 60 times 80 is 4 thousand and 800. | ||
| + | |style="text-align:right;"|ואם תרצה לדעת כמה שוה השני אמו' ס' פעמ' פ' ד' אלפים ות"ת | ||
| + | |- | ||
| + | | | ||
| + | :Divide it by 154; the result is 31 pešuṭim and 26 parts of 154. | ||
| + | :<math>\scriptstyle{\color{blue}{a_2=\frac{60\sdot80}{154}=\frac{4800}{154}=31+\frac{26}{154}}}</math> | ||
| + | |style="text-align:right;"|חלקם בקנ"ד יבואו ל"א פשוטי' וכ"ו חלקים מקנ"ד | ||
| + | |- | ||
| + | | | ||
| + | :<span style=color:green>'''Rule of Three:'''</span> To know how much the third is worth, say: 30 times 80 is 2 thousand and 400. | ||
| + | |style="text-align:right;"|ולדעת חלק השלישי אמו' ל' פעמי' פ' ב' אלפים ות‫' | ||
|- | |- | ||
| | | | ||
| − | : | + | :Divide it by 154; the result is 15 pešuṭim and 90 parts of 154. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{a_3=\frac{30\sdot80}{154}=\frac{2400}{154}=15+\frac{90}{154}}}</math> |
| − | חלקם בקנ"ד יבואו | + | |style="text-align:right;"|חלקם בקנ"ד יבואו ט"ו פשוטי' וצ' חלקים מקנ"ד |
|- | |- | ||
| | | | ||
| − | : | + | :<span style=color:green>'''Rule of Three:'''</span> To know how much the fourth is worth, say: 24 times 80 is a thousand and 920. |
| − | |style="text-align:right;"|ולדעת חלק | + | |style="text-align:right;"|ולדעת חלק הרביעי אמ' כ"ד פעמי' פ' הם אלף ותתק"כ |
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :Divide it by 154; the result is 12 and 72 parts of 154. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{a_4=\frac{24\sdot80}{154}=\frac{1920}{154}=12+\frac{72}{154}}}</math> |
| − | חלקם בקנ"ד יבואו י"ב וע"ב חלקים מקנ"ד | + | |style="text-align:right;"|חלקם בקנ"ד יבואו י"ב וע"ב חלקים מקנ"ד |
|- | |- | ||
| | | | ||
| − | : | + | :Do the same if the four coins are worth all in all 100 pešuṭim: |
|style="text-align:right;"|וכן תעש' ג"כ אם יהיו שוים ד' המטבעו' האילו ק' פשוטי' בין כולם | |style="text-align:right;"|וכן תעש' ג"כ אם יהיו שוים ד' המטבעו' האילו ק' פשוטי' בין כולם | ||
|- | |- | ||
| | | | ||
| − | : | + | :<span style=color:green>'''Rule of Three:'''</span> To know how much is the first, say: 40 times 100; then, divide it by 154. |
| + | :<math>\scriptstyle{\color{blue}{a_1=\frac{40\sdot100}{154}}}</math> | ||
|style="text-align:right;"|לדעת הראשו' אמו' מ' פעמ' ק' וחלקם בקנ"ד | |style="text-align:right;"|לדעת הראשו' אמו' מ' פעמ' ק' וחלקם בקנ"ד | ||
|- | |- | ||
| | | | ||
| − | : | + | :<span style=color:green>'''Rule of Three:'''</span> To know how much is the second, say: 60 times 100; then, divide it by 154. |
| + | :<math>\scriptstyle{\color{blue}{a_2=\frac{60\sdot100}{154}}}</math> | ||
|style="text-align:right;"|ולדעת השני אמו' ס' פעמ' ק' וחלקם בקנ"ד | |style="text-align:right;"|ולדעת השני אמו' ס' פעמ' ק' וחלקם בקנ"ד | ||
|- | |- | ||
| | | | ||
| − | : | + | :<span style=color:green>'''Rule of Three:'''</span> To know how much is the third, say: 30 times 100; then, divide it by 154. |
| + | :<math>\scriptstyle{\color{blue}{a_3=\frac{30\sdot100}{154}}}</math> | ||
|style="text-align:right;"|ולדעת השלישי אמו' ל' פעמ' ק' וחלקם בקנ"ד | |style="text-align:right;"|ולדעת השלישי אמו' ל' פעמ' ק' וחלקם בקנ"ד | ||
|- | |- | ||
| | | | ||
| − | : | + | :<span style=color:green>'''Rule of Three:'''</span> To know how much is the fourth, say: 24 times 100; then, divide it by 154. |
| + | :<math>\scriptstyle{\color{blue}{a_4=\frac{24\sdot100}{154}}}</math> | ||
|style="text-align:right;"|ולדעת הרביעי אמו' כ"ד פעמ' ק' וחלקם בקנ"ד | |style="text-align:right;"|ולדעת הרביעי אמו' כ"ד פעמ' ק' וחלקם בקנ"ד | ||
|- | |- | ||
| | | | ||
| + | :The same for any number you want: find it this way. | ||
|style="text-align:right;"|וכן לכל חשבו' שתרצה תפוש אותו על זה הדרך | |style="text-align:right;"|וכן לכל חשבו' שתרצה תפוש אותו על זה הדרך | ||
|- | |- | ||
| Line 5,725: | Line 7,400: | ||
*{{#annot:⅓·¼·⅕|668|SN3Z}}Who has the third of the quarter of the fifth | *{{#annot:⅓·¼·⅕|668|SN3Z}}Who has the third of the quarter of the fifth | ||
:<math>\scriptstyle\frac{1}{3}\sdot\frac{1}{4}\sdot\frac{1}{5}</math> | :<math>\scriptstyle\frac{1}{3}\sdot\frac{1}{4}\sdot\frac{1}{5}</math> | ||
| − | |style="text-align:right;"|מי שיש לו השליש מן הרביע מן החומש{{#annotend:SN3Z}} | + | |style="text-align:right;"|‫<ref>MS Ithaca om.</ref>מי שיש לו השליש מן הרביע מן החומש{{#annotend:SN3Z}} |
|- | |- | ||
| | | | ||
| + | :It has one part of 60. | ||
:<math>\scriptstyle{\color{blue}{\frac{1}{3}\sdot\frac{1}{4}\sdot\frac{1}{5}=\frac{1}{60}}}</math> | :<math>\scriptstyle{\color{blue}{\frac{1}{3}\sdot\frac{1}{4}\sdot\frac{1}{5}=\frac{1}{60}}}</math> | ||
|style="text-align:right;"|יש לו א' חלק מס‫' | |style="text-align:right;"|יש לו א' חלק מס‫' | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{ | + | :Because the fifth of 60 is 12; the quarter of 12 is 3; and the third of 3 is 1. |
| − | |style="text-align:right;"|כי החומש מס' הוא י"ב | + | :<math>\scriptstyle{\color{blue}{\frac{1}{3}\sdot\frac{1}{4}\sdot\left(\frac{1}{5}\sdot60\right)=\frac{1}{3}\sdot\left(\frac{1}{4}\sdot12\right)=\frac{1}{3}\sdot3=1}}</math> |
| − | והרביע מי"ב הוא ג | + | |style="text-align:right;"|כי החומש מס' הוא י"ב והרביע מי"ב הוא ג' והשליש מג' הוא א‫' |
| − | והשליש מג' הוא א‫' | ||
|- | |- | ||
| | | | ||
| Line 5,743: | Line 7,418: | ||
|- | |- | ||
| | | | ||
| + | :It has 1 part of 360. | ||
:<math>\scriptstyle{\color{blue}{\frac{1}{3}\sdot\frac{1}{4}\sdot\frac{1}{5}\sdot\frac{1}{6}=\frac{1}{360}}}</math> | :<math>\scriptstyle{\color{blue}{\frac{1}{3}\sdot\frac{1}{4}\sdot\frac{1}{5}\sdot\frac{1}{6}=\frac{1}{360}}}</math> | ||
|style="text-align:right;"|יש לו א' חלק מש"ס | |style="text-align:right;"|יש לו א' חלק מש"ס | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{6\sdot60=360}}</math> | + | :360 results from 6 times 60. |
| + | :<math>\scriptstyle{\color{blue}{6\sdot60=360}}</math> | ||
|style="text-align:right;"|וש"ס יוצא מו' פעמ' ס‫' | |style="text-align:right;"|וש"ס יוצא מו' פעמ' ס‫' | ||
|- | |- | ||
| Line 5,756: | Line 7,433: | ||
|- | |- | ||
| | | | ||
| + | :It has one part of 2 thousand and 520. | ||
:<math>\scriptstyle{\color{blue}{\frac{1}{3}\sdot\frac{1}{4}\sdot\frac{1}{5}\sdot\frac{1}{6}\sdot\frac{1}{7}=\frac{1}{2520}}}</math> | :<math>\scriptstyle{\color{blue}{\frac{1}{3}\sdot\frac{1}{4}\sdot\frac{1}{5}\sdot\frac{1}{6}\sdot\frac{1}{7}=\frac{1}{2520}}}</math> | ||
|style="text-align:right;"|יש לו א' חלק מב' אלפי' ותק"כ | |style="text-align:right;"|יש לו א' חלק מב' אלפי' ותק"כ | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{7\sdot360=2520}}</math> | + | :It results from 7 times 360. |
| + | :<math>\scriptstyle{\color{blue}{7\sdot360=2520}}</math> | ||
|style="text-align:right;"|וזה יוצא מן ז' פעמ' ש"ס | |style="text-align:right;"|וזה יוצא מן ז' פעמ' ש"ס | ||
|- | |- | ||
| Line 5,769: | Line 7,448: | ||
|- | |- | ||
| | | | ||
| + | :It has one part of 20 thousand and 160. | ||
:<math>\scriptstyle{\color{blue}{\frac{1}{3}\sdot\frac{1}{4}\sdot\frac{1}{5}\sdot\frac{1}{6}\sdot\frac{1}{7}\sdot\frac{1}{8}=\frac{1}{20160}}}</math> | :<math>\scriptstyle{\color{blue}{\frac{1}{3}\sdot\frac{1}{4}\sdot\frac{1}{5}\sdot\frac{1}{6}\sdot\frac{1}{7}\sdot\frac{1}{8}=\frac{1}{20160}}}</math> | ||
|style="text-align:right;"|יש לו א' חלק מכ' אלפי' וק"ס | |style="text-align:right;"|יש לו א' חלק מכ' אלפי' וק"ס | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{8\sdot2520=20160}}</math> | + | :It results from 8 times 2 thousand and 520. |
| + | :<math>\scriptstyle{\color{blue}{8\sdot2520=20160}}</math> | ||
|style="text-align:right;"|וזה יוצא מח' פעמ' ב' אלפים ותק"כ | |style="text-align:right;"|וזה יוצא מח' פעמ' ב' אלפים ותק"כ | ||
|- | |- | ||
| Line 5,782: | Line 7,463: | ||
|- | |- | ||
| | | | ||
| + | :It has one part of 181 thousand and 4[4]0. | ||
:<math>\scriptstyle{\color{blue}{\frac{1}{3}\sdot\frac{1}{4}\sdot\frac{1}{5}\sdot\frac{1}{6}\sdot\frac{1}{7}\sdot\frac{1}{8}\sdot\frac{1}{9}=\frac{1}{1814{\color{red}{40}}}}}</math> | :<math>\scriptstyle{\color{blue}{\frac{1}{3}\sdot\frac{1}{4}\sdot\frac{1}{5}\sdot\frac{1}{6}\sdot\frac{1}{7}\sdot\frac{1}{8}\sdot\frac{1}{9}=\frac{1}{1814{\color{red}{40}}}}}</math> | ||
| − | |style="text-align:right;"|יש לו א' חלק מקפ"א אלפי' ות" | + | |style="text-align:right;"|יש לו א' חלק מקפ"א אלפי' ות"כ |
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{9\sdot20160=1814{\color{red}{40}}}}</math> | + | :It results from 9 times 20 thousand and 160. |
| + | :<math>\scriptstyle{\color{blue}{9\sdot20160=1814{\color{red}{40}}}}</math> | ||
|style="text-align:right;"|וזה יוצא מט' פעמ' כ' אלפים וק"ס | |style="text-align:right;"|וזה יוצא מט' פעמ' כ' אלפים וק"ס | ||
|- | |- | ||
| Line 5,795: | Line 7,478: | ||
|- | |- | ||
| | | | ||
| + | :It has one part of a thousand of thousands, 814 thousand and [4]00. | ||
:<math>\scriptstyle{\color{blue}{\frac{1}{3}\sdot\frac{1}{4}\sdot\frac{1}{5}\sdot\frac{1}{6}\sdot\frac{1}{7}\sdot\frac{1}{8}\sdot\frac{1}{9}\sdot\frac{1}{10}=\frac{1}{1814{\color{red}{400}}}}}</math> | :<math>\scriptstyle{\color{blue}{\frac{1}{3}\sdot\frac{1}{4}\sdot\frac{1}{5}\sdot\frac{1}{6}\sdot\frac{1}{7}\sdot\frac{1}{8}\sdot\frac{1}{9}\sdot\frac{1}{10}=\frac{1}{1814{\color{red}{400}}}}}</math> | ||
| − | |style="text-align:right;"|יש לו א' חלק מאלף אלפים ותתי"ד אלפי | + | |style="text-align:right;"|יש לו א' חלק מאלף אלפים ותתי"ד אלפי' ור‫' |
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{10\sdot1814{\color{red}{40}}=1814{\color{red}{400}}}}</math> | + | :It results from 10 times 181 thousand and 4[4]0. |
| − | |style="text-align:right;"|וזה יוצא מי' פעמ' קפ"א אלפי' ות" | + | :<math>\scriptstyle{\color{blue}{10\sdot1814{\color{red}{40}}=1814{\color{red}{400}}}}</math> |
| + | |style="text-align:right;"|וזה יוצא מי' פעמ' קפ"א אלפי' ות"כ | ||
|- | |- | ||
| | | | ||
| − | === Pricing Problems === | + | === <span style=color:green>Pricing Problems</span> === |
| | | | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:oil|629|HxPU}} | + | *{{#annot:oil|629|HxPU}}One thousand of oil are equal to 40 mitri(?). |
| − | :If | + | :If the thousand is worth 27 liṭra and 5 dinar, how much is one mitro(?) worth? |
:<math>\scriptstyle\frac{\left[27\sdot\left(20\sdot12\right)\right]+\left(5\sdot12\right)}{40}=\frac{X}{1}</math> | :<math>\scriptstyle\frac{\left[27\sdot\left(20\sdot12\right)\right]+\left(5\sdot12\right)}{40}=\frac{X}{1}</math> | ||
| − | |style="text-align:right;"|האלף של שמן הוא מ' מיטרי א‫'<br> | + | |style="text-align:right;"|‫<ref>MS Ithaca om.</ref>האלף של שמן הוא מ' מיטרי א‫'<br> |
אם האלף שווה כ"ז ליט' וה' די' כמה יבא המיטרו{{#annotend:HxPU}} | אם האלף שווה כ"ז ליט' וה' די' כמה יבא המיטרו{{#annotend:HxPU}} | ||
|- | |- | ||
| | | | ||
| − | : | + | :Take 6 pešuṭim for each liṭra; and a fifth plus a half [of a fifth], which is 3-tenths, for each dinar. |
| − | + | :<math>\scriptstyle{\color{OliveGreen}{X=\left(27\sdot6\right)+\left(5\sdot\frac{3}{10}\right)=\left(27\sdot6\right)+\left[5\sdot\left[\frac{1}{5}+\left(\frac{1}{2}\sdot\frac{1}{5}\right)\right]\right]}}</math> | |
| − | + | |style="text-align:right;"|תפוש מכל ליט' ו' פשו' ומכל די' א' חומש וחצי שהם ג' עשירייו‫' | |
| − | |style="text-align:right;"|תפוש מכל ליט' ו' פשו | ||
| − | ומכל די' א' חומש וחצי שהם ג' עשירייו‫' | ||
|- | |- | ||
| | | | ||
| Line 5,829: | Line 7,512: | ||
|- | |- | ||
| | | | ||
| − | : | + | :Take 1 pašuṭ and a fifth for each liṭra. |
| − | + | :<math>\scriptstyle{\color{OliveGreen}{X=25\sdot\left(1+\frac{1}{5}\right)}}</math> | |
|style="text-align:right;"|תפוש מכל ליט' א' פשוט וחומש | |style="text-align:right;"|תפוש מכל ליט' א' פשוט וחומש | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | === Divide a Quantity Problems === | + | === <span style=color:green>Divide a Quantity Problems</span> === |
| | | | ||
|- | |- | ||
| | | | ||
| − | *{{#annot:money|645|o6n6}}Dividing 9 dinar to a half, a third, and a ninth | + | *{{#annot:money|645|o6n6}}Dividing 9 dinar to a half, a third, and a ninth. |
:<math>\scriptstyle\frac{1}{2}X+\frac{1}{3}X+\frac{1}{9}X=9</math> | :<math>\scriptstyle\frac{1}{2}X+\frac{1}{3}X+\frac{1}{9}X=9</math> | ||
| − | |style="text-align:right;"|לחלק ט' דינרי' לחצי לשליש ולתשיע{{#annotend:o6n6}} | + | |style="text-align:right;"|<big>לחלק ט' דינרי' לחצי לשליש ולתשיע</big>{{#annotend:o6n6}} |
|- | |- | ||
| | | | ||
| − | + | :<span style=color:green>'''False Position - denominator:'''</span> Say: a half, a third, and a ninth are found in 18. | |
| − | + | |style="text-align:right;"|אמור חצי ושליש ותשיע ימצאו בי"ח | |
| − | |style="text-align:right;"|אמור חצי ושליש ותשיע ימצאו בי"ח | ||
|- | |- | ||
| | | | ||
| − | : | + | :The half is 9; the third is 6; the ninth is 2; their sum is 17 and this is the denominator. |
| − | + | |style="text-align:right;"|החצי ט' השליש ו' התשיע ב' וכללם י"ז והוא המחלק | |
| − | |style="text-align:right;"| | ||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |colspan="2" | |
| − | + | :<math>\scriptstyle{\color{blue}{\left(\frac{1}{2}\sdot18\right)+\left(\frac{1}{3}\sdot18\right)+\left(\frac{1}{9}\sdot18\right)=9+6+2=17}}</math> | |
|- | |- | ||
| | | | ||
| − | :: | + | :To know the portion of the one who has a half: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ולדעת חלק אותו שיש לו החצי |
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | :: | + | :<span style=color:green>'''Rule of Three:'''</span> Say: [a half] of 18 is 9. Multiply it by the number of dinar, which is 9, and say: 9 times 9 is 81. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אמור מי"ח הוא ט' ותרבה אותם עם סך הדינרי' שהם ט' ואמור ט' פעמים ט' הם פ"א |
|- | |- | ||
| | | | ||
| − | : | + | :Divide it by 17; it is [4] dinar and 13 parts of 17 of one dinar. |
| − | + | |style="text-align:right;"|חלקם בי"ז יהיו י"ד דינרי' וי"ג חלקים מי"ז מן הדינר | |
| − | |style="text-align:right;"| | ||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | |colspan="2" | |
| − | + | :<math>\scriptstyle{\color{blue}{\frac{1}{2}X=\frac{\left(\frac{1}{2}\sdot18\right)\sdot9}{17}=\frac{9\sdot9}{17}=\frac{81}{17}=4+\frac{13}{17}}}</math> | |
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :We should divide the dinar into 17 parts; so one part is 12 parts of 17 of one pašuṭ. |
| − | + | |style="text-align:right;"|נצטרך אם כן לחלק הדינר בי"ז חלקים ויהיה החלק י"ב חלקי' מי"ז מן הפשוט | |
| − | |||
| − | |style="text-align:right;"| | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :If we want to know how much are 13 parts of 17 of a dinar: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<big>ואם נרצה</big> לדעת הי"ג חלקים מי"ז מן הדינר כמה הם |
|- | |- | ||
| | | | ||
| − | + | :We say: 12 times 13 is 156. | |
| − | : | + | |style="text-align:right;"|נאמר <sup>י"ב</sup>י"ג פעמים י"ג הם קנ"ו |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | : | + | :Divide it by 17; it is 9 pešuṭim and 3 parts of 17. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|חלקם בי"ז יהיו ט"פ וג' חלקי' מי"ז |
|- | |- | ||
| − | | | + | |colspan="2" | |
| − | + | :<math>\scriptstyle{\color{blue}{\frac{13\sdot12}{17}=\frac{156}{17}=9+\frac{3}{17}}}</math> | |
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :Therefore, the owner of the a half has 4 dinar, 9 pešuṭim and 3 parts of 17 of one pašuṭ. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|הרי אותו שיש לו החצי יש לו לחלקו ד' דינרי' וט"פ וג' חלקי' מי"ז מהפשוט |
| − | |||
|- | |- | ||
| | | | ||
| − | :: | + | :To know the portion of the one who has a third: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<big>ולדעת חלק</big> אותו שיש לו השליש |
|- | |- | ||
| | | | ||
| − | : | + | :<span style=color:green>'''Rule of Three:'''</span> Multiply 6, which is a third of 18, by 9, which is the number of dinar, and say: 6 times 9 is 54. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|תרבה ו' שהוא השליש מי"ח עם ט' שהוא סכום הדינרי' ואמור ו' פעמי' ט' נ"ד‫<ref>ט' נ"ד: MS Ithaca om.</ref> |
|- | |- | ||
| | | | ||
| − | : | + | :Divide it by 17 [parts] of one dinar; it is 3 dinar and 3 parts of 17 of one dinar. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|חלקם בי"ז מהדינר יבואו ג' די' וג' חלקים מי"ז מן הדי‫'‫<ref>יבואו ג'... מן הדי': MS Ithaca om.</ref> |
|- | |- | ||
| − | | | + | |colspan="2" | |
| − | : | + | :<math>\scriptstyle{\color{blue}{\frac{1}{3}X=\frac{\left(\frac{1}{3}\sdot18\right)\sdot9}{17}=\frac{6\sdot9}{17}=\frac{54}{17}=3+\frac{3}{17}}}</math> |
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :To know how much are 3 parts of 17 of one dinar: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ולדעת כמה הם אילו הג' חלקים מי"ז מהדינר |
|- | |- | ||
| | | | ||
| − | :: | + | :Say: 3 times 12 is 36. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אמור ג' פעמים י"ב ל"ו |
|- | |- | ||
| | | | ||
| − | : | + | :Divide it by 17; the result is 2 pešuṭim and 2 parts of 17 of one pašuṭ. |
| − | + | |style="text-align:right;"|חלקם בי"ז יבאו ב"פ וב' חלקים מי"ז מהפשוט | |
| − | |style="text-align:right;"| | ||
|- | |- | ||
| − | |} | + | |colspan="2" | |
| − | { | + | :<math>\scriptstyle{\color{blue}{\frac{3\sdot12}{17}=\frac{36}{17}=2+\frac{2}{17}}}</math> |
|- | |- | ||
| | | | ||
| − | + | :To know the portion of the one who has a ninth: | |
| − | = | + | |style="text-align:right;"|‫<ref>45v</ref><big>ולדעת חלק אותו שיש לו התשיע</big> |
| − | + | |- | |
| | | | ||
| + | :<span style=color:green>'''Rule of Three:'''</span> Multiply 2, which is a ninth of 18, by 9, which is the number of dinar, and say: 2 times 9 is 18. | ||
| + | |style="text-align:right;"|<big>תרבה ב' שהוא התשיע</big> מי"ח עם הדינר שהוא סך הדינר <s>מי"ח עם הדינר שהוא סך הדינר</s> ואמור ב' פע' ט' י"ח | ||
|- | |- | ||
| | | | ||
| − | + | :Divide it by 17; it is 1 dinar and 1 part of 17 of one dinar, which is 12 parts of 17 of one pašuṭ. | |
| − | + | |style="text-align:right;"|חלקם בי"ז יהיו א' דינר וא' חלק מי"ז מהדינר שהוא י"ב חלקי' מי"ז <sup>מ</sup>הפשוט | |
| − | + | |- | |
| − | + | | colspan="2"| | |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{\frac{1}{9}X=\frac{\left(\frac{1}{9}\sdot18\right)\sdot9}{17}=\frac{2\sdot9}{17}=\frac{18}{17}=1+\frac{1}{17}=1+\frac{\frac{12}{17}}{12}}}</math> |
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :Therefore, the owner of a ninth has 1 dinar and 12 parts of 17 of one pašuṭ. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|הרי שבעל התשיע יש לו א' דינר וי"ב חלקי' מי"ז מהפשוט |
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle | + | *{{#annot:money|645|998G}}Dividing 9 liṭra to a half, a third, and a ninth. |
| − | + | :<math>\scriptstyle\frac{1}{2}X+\frac{1}{3}X+\frac{1}{9}X=9</math> | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ולחלק ט' ליט' לחצי לשליש ולתשיע{{#annotend:998G}} |
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{ | + | :The owner of the half has 4 liṭra and 13 parts of 17 of one liṭra. |
| − | \frac | + | :<math>\scriptstyle{\color{blue}{\frac{1}{2}X=4+\frac{13}{17}}}</math> |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|יהיה א"כ לבעל החצי ד' ליטרי' וי"ג חלקי' מי"ז מהלי‫' |
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | + | :To know how much are the 13 parts of 17 of [one] liṭra: | |
| − | : | + | |style="text-align:right;"|<big>ולדעת</big> כמה הם הי"ג חלקי' מהי"ז מן הליטרי‫' |
| − | |||
| − | |style="text-align:right;"| | ||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :Divide one liṭra, which are 240 pešuṭim, into 17 parts; each part is 14 pešuṭim and 2 parts of 17 of one pašuṭ. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{\frac{240}{17}=14+\frac{2}{17}}}</math> |
| + | |style="text-align:right;"|חלק הליט' שהו' ר"מ פשיטי' בי"ז חלקים יהיה כל חלק יד"פ וב' חלקי' מי"ז מן הפשוט | ||
|- | |- | ||
| | | | ||
| − | :: | + | :If you want to know the 13 parts of 17 of one liṭra: |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|<big>ואם תרצה</big> לדעת הי"ג חלקי' מי"ז מן הליטרא |
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\ | + | :Say: 13 times 14 pešuṭim and 2 parts of 17 are 15 dinar, 3 pešuṭim and 9 parts of 17. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{13\sdot\left(14+\frac{2}{17}\right)=\left(15\sdot12\right)+3+\frac{9}{17}}}</math> |
| − | + | |style="text-align:right;"|אמור י"ג פעמים יד"פ וב' חלקי' מי"ז הם ט"ו דינרים וג"פ וט' חלקי' מי"ז | |
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :So, the owner of the half has 4 liṭra, 15 dinar, 3 pešuṭim and [9] parts of 17 of one pašuṭ. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|הרי שבעל החצי יש לו ד' ליטרי' וט"ו דינרי' וג"פ וט"ו חלקי' מי"ז מהפשוט |
|- | |- | ||
| | | | ||
| − | : | + | :The owner of the third has 3 liṭra and 13 parts of 17 of one liṭra. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{\frac{1}{3}X=3+\frac{3}{17}}}</math> |
| + | |style="text-align:right;"|ואותו שיש לו השליש יש לו ג' ליטרי' וג' חלקים מי"ז <s>מהפשוט</s> מהליט‫' | ||
|- | |- | ||
| | | | ||
| − | + | :The 3 parts of 17 of one liṭra are 3 times 14 pešuṭim and 3 times 2 parts of 17 of one pašuṭ. | |
| − | : | + | |style="text-align:right;"|והג' חלקים מי"ז מן הליט'‫<ref>והג'... מן הליט': MS Ithaca om.</ref> הם ג' פעמים יד"פ וג' פעמים ב' חלקים מי"ז מהפשוט |
| − | : | + | |- |
| − | + | | colspan="2"| | |
| − | :<math>\scriptstyle\frac{\left( | + | :<math>\scriptstyle{\color{blue}{\frac{3}{17}=\frac{3\sdot\left(14+\frac{2}{17}\right)}{20\sdot12}}}</math> |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :The total are 3 liṭra, 3 dinar, 6 pešuṭim and 6 parts of 17 of one pašuṭ. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|שהם בין הכל ג' ליטרי' וג' דינרי' וו' פשיטי' וו' חלקי' מי"ז מהפשוט |
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{ | + | :The owner of the ninth has 1 liṭra and 1 part of 17 of one liṭra. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{\frac{1}{9}X=1+\frac{1}{17}}}</math> |
| − | + | |style="text-align:right;"|ואותו שיש לו התשיע יש לו א' ליטר' וא' חלק מי"ז מהליט‫' | |
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :One part of 17 of one liṭra is 14 pešuṭim and 2 parts of 17 of one pašuṭ. |
| − | |style="text-align:right;"| | + | :<math>\scriptstyle{\color{blue}{\frac{1}{17}=\frac{14+\frac{2}{17}}{20\sdot12}}}</math> |
| + | |style="text-align:right;"|וא' חלק מי"ז הליט' הוא יד"פ וב' חלקי' מי"ז מהפשוט | ||
|- | |- | ||
| | | | ||
| − | ::<math>\scriptstyle{\color{blue}{\ | + | :<span style=color:green>'''Check:'''</span> If you sum up all the portions, they are exactly 9 liṭra. |
| − | + | |style="text-align:right;"|ואם תקבץ כל החלקי' יהיו ט' ליטרין ממש | |
| − | + | |- | |
| − | + | | colspan="2"| | |
| − | + | :<math>\scriptstyle{\color{blue}{\frac{1}{2}X+\frac{1}{3}X+\frac{1}{9}X=\left(4+\frac{15}{20}+\frac{3+\frac{9}{17}}{20\sdot12}\right)+\left(3+\frac{3}{20}+\frac{6+\frac{6}{17}}{20\sdot12}\right)+\left(1+\frac{14+\frac{2}{17}}{20\sdot12}\right)=9}}</math> | |
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | + | ||
| − | + | === <span style=color:green>Guessing a chosen number (MS Verona)</span> === | |
| − | + | ||
| − | + | | | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | *{{#annot:chosen number|667|DLI4}}To find [the number] that a man has chosen, tell the man to choose [a number] as he pleases and double it twice, or three times, or four, as you wish to ask him. |
| − | |style="text-align:right;"| | + | :Then tell him to divide [the product] by [the original number] that he thought of at first. |
| + | :You can figure it out by doing the same with 1, and your remainder is the same as his remainder no less and no more | ||
| + | :<math>\scriptstyle\frac{x\sdot2^n}{x}=2^n</math> | ||
| + | |style="text-align:right;"|לדעת מה יחשוב האדם אמור לאדם שיחשוב כרצונו ויכפלם פעמים או שלוש או ארבע כמו שתרצה לומ' לו<br> | ||
| + | א"כ תאמ' לו שיחלקם כפי המחשבה הראשונה שחשב<br> | ||
| + | וזה תוכל לדעת בעשותך גם אתה החשבון על אחד והנשאר בידך הוא הנשאר בידו לא פחות ולא יתר{{#annotend:DLI4}} | ||
|- | |- | ||
| | | | ||
| − | :: | + | :Example: if he thought of the number 4 |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|דמיון זה כאלו חשב ד‫' |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | + | :Double it once; it is 8. | |
| − | + | |style="text-align:right;"|כפול אותם פעם אחת יהיו ח‫' | |
| − | |||
| − | |style="text-align:right;"| | ||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :Double it a second time; it is 16. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כפול אותם שנית יהיו י"ו |
|- | |- | ||
| | | | ||
| − | : | + | :Double it a third time; it is 32. |
| − | |style="text-align:right;"|כפול אותם | + | |style="text-align:right;"|כפול אותם שלישית יהיה ל"ב |
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :Double it a fourth time; it is 64. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כפול אותם רביעית יהיו ס"ד |
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | + | :Divide it by the 4 he thought of at first; 16 remains. | |
| − | + | |style="text-align:right;"|חלקם על ד' שחשב בראשונה נשארו י"ו | |
| − | : | + | |- |
| − | + | | colspan="2"| | |
| − | + | :<math>\scriptstyle{\color{blue}{\frac{4\sdot2^4}{4}=\frac{\left[\left[\left(4\sdot2\right)\sdot2\right]\sdot2\right]\sdot2}{4}=\frac{\left[\left(8\sdot2\right)\sdot2\right]\sdot2}{4}=\frac{\left(16\sdot2\right)\sdot2}{4}=\frac{32\sdot2}{4}=\frac{64}{4}=16}}</math> | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | :<math>\scriptstyle | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| − | | | + | | |
| − | |style="text-align:right;"| | + | :You think of 1. |
| + | |style="text-align:right;"|ואתה חשבת א‫' | ||
|- | |- | ||
| − | |||
| | | | ||
| + | :You double it once; it is 2. | ||
| + | |style="text-align:right;"|כפלת אותו פעם אחת יהיו ב‫' | ||
|- | |- | ||
| | | | ||
| − | + | :You double 2; it is 4. | |
| − | + | |style="text-align:right;"|כפלת ב' יהיו ד‫' | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |style="text-align:right;"| | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :You double 4; it is 8. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כפלת ד' יהיו ח‫' |
|- | |- | ||
| | | | ||
| − | : | + | :You double 8; it is 16. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כפול ח' יהיו י"ו |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | :Divide it by 1; it is 16 as what he [got]. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|חלקם על א' יהיו כמוהו י"ו בשוה |
|- | |- | ||
| − | | | + | | colspan="2"| |
| − | : | + | :<math>\scriptstyle{\color{blue}{\frac{1\sdot2^4}{1}=\frac{\left[\left[\left(1\sdot2\right)\sdot2\right]\sdot2\right]\sdot2}{1}=\frac{\left[\left(2\sdot2\right)\sdot2\right]\sdot2}{1}=\frac{\left(4\sdot2\right)\sdot2}{1}=\frac{8\sdot2}{1}=\frac{16}{1}=16}}</math> |
| − | |||
|- | |- | ||
| | | | ||
| − | : | + | *{{#annot:fish price|667|K5o4}}Tell [a man] to think of [a certain amount of] dinar, then add so and so pešuṭim for each dinar, double the sum, and buy fishes with [the result], paying for each the same number [of pešuṭim] added for each dinar. |
| − | |style="text-align:right;"| | + | :You will be able to know [the chosen number] by calculating to yourself [using the same procedure] with 12. |
| + | |style="text-align:right;"|אחר אמו' שיחשוב דינרי' ויוסיף עליו כך וכך פשו' בכל לי' ויכפלם ויקנה מהם דגים שיתן לכל אחד כמספר התוספת שהוסיף בין כל הלי‫'<br> | ||
| + | וזה תוכל לדעת שתעשה החשבון בינך לבינך על י"ב{{#annotend:K5o4}} | ||
|- | |- | ||
| − | |} | + | | colspan="2"| |
| − | { | + | :<span style=color:green>the number of fishes that can be bought =</span> <math>\scriptstyle{\color{OliveGreen}{\frac{\left[12x+\left(a\sdot x\right)\right]\sdot2}{a}}}</math> |
|- | |- | ||
| | | | ||
| − | + | :*Example: he thought of 3 dinar and we told him to add 3 pešuṭim for each dinar. | |
| − | + | |style="text-align:right;"|דמיון זה שחשב ג' לי' ואמרנו לו שיוסיף בכל די' ג' פ‫' | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | |style="text-align:right;"| | + | ::The total is 45 pešuṭim. |
| + | |style="text-align:right;"|והנה עלו כל הפשו' מ"ה | ||
|- | |- | ||
| | | | ||
| − | + | ::We tell him to double them; the result is 90 pešuṭim. | |
| − | + | |style="text-align:right;"|עו' אמרנו לו שיכפלם ועלו צ' פשו‫' | |
| − | |||
| − | |style="text-align:right;"| | ||
|- | |- | ||
| | | | ||
| − | + | ::When we tell him to buy fishes with them and he pays 3 pešuṭim for each, as the number we told him to add, he buys 30 fishes. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכאשר אמרנו לו שיקנה מהם דגים ויתן בכל אחד ג' פשו' כמספר התוספת שאמרנו לו שיוסיף הנה קנה ל' דגים |
|- | |- | ||
| − | | | + | | colspan="2"| |
| − | + | ::<math>\scriptstyle{\color{blue}{\frac{\left[\left(12\sdot3\right)+\left(3\sdot3\right)\right]\sdot2}{3}=\frac{45\sdot2}{3}=\frac{90}{3}=30}}</math> | |
| − | |||
|- | |- | ||
| | | | ||
| − | + | ::When you calculate it for your self with 12, you say that he was thinking of one dinar, which is 12 pešuṭim. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והנה כשתעשה החשבון בינך לבינך על י"ב שתאמר שחשב א' לי' והוא י"ב פשו‫' |
|- | |- | ||
| − | | | + | | colspan="2"| |
| − | + | ::<math>\scriptstyle{\color{blue}{\left[\left(12\sdot1\right)+\left(3\sdot1\right)\right]\sdot2=\left(12+3\right)\sdot2=15\sdot2=30}}</math> | |
| − | |||
|- | |- | ||
| | | | ||
| − | + | ::When he adds 3 pešuṭim to them, they are 15. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|הנה כאשר הוסיף ג' פשו' עליהם ועלו ט"ו |
|- | |- | ||
| | | | ||
| − | + | ::Double them; they are 30. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכפלם ועלו ל‫' |
|- | |- | ||
| | | | ||
| − | + | ::He bought fishes with them and paid 3 pešuṭim for each, which is the number of the addition; the result is 10 fishes. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וקנה מהם דגים ונתן בכל אחד ג' פשו' שהוא מספר התוספת ועלו הדגים י‫' |
|- | |- | ||
| | | | ||
| − | + | ::Hence, you know that if he says that he bought 10 fishes, you can deduce that he was thinking of one dinar. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|הנה תידע שאם יאמ' שקנה י' דגים תידע שחשב א' לי‫' |
|- | |- | ||
| | | | ||
| − | + | ::If he said that he bought 20 fishes, you can deduce that he was thinking of 2 dinar. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכן אם אמר שקנה כ דגים תידע שחשב ב' לי‫' |
|- | |- | ||
| | | | ||
| − | * | + | *{{#annot:chosen number|667|T8nJ}}Tell a man to think [of a number], add twice of it, then halve [the sum], and if there is a half [in the result] he should consider it as a whole [i.e. round it up]. |
| − | |style="text-align:right;"| | + | :Then he should add to half the sum twice of it, and cast out the nines. |
| + | :[The chosen number] is found by taking two for each nine and [the result] is the number he thought of, if there is no remainder [from casting out the nines]. | ||
| + | :If there is a remainder [from casting out the nines] it is 6 […] and for the remainder take one [and add it to the previous result]. | ||
| + | :<math>\scriptstyle\frac{\left(2\sdot\frac{2x+x}{2}\right)+\frac{2x+x}{2}}{9}\sdot2=x</math> | ||
| + | |style="text-align:right;"|אחר אמור לו שיחשוב ויכפלם פי שנים ויחלקם לחצי אם יש שם חצי יחשבהו שלם<br> | ||
| + | ויכפול זה החצי פי שנים וישליכם ט' ט‫'<br> | ||
| + | וזה יודע שתקח לכל ט' שיש בידו ב' וכן חשב כשלא ישאר בידו כלום<br> | ||
| + | ואם ישארו בידו כלום יהיו ו' ואם לא יגיעו לכל ט' חשב א' והם ו‫'<br> | ||
| + | ואם היו יותר מט' ונשארו בידו תקח המספר הידוע לכל ט' ובעבו' הנשארים תקח א‫'{{#annotend:T8nJ}} | ||
|- | |- | ||
| | | | ||
| − | * | + | :*Example: If he thought of 20 |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|דמיון זה כאילו חשב כ‫' |
|- | |- | ||
| | | | ||
| − | + | ::We tell him to duplicate it twice; the result is 60. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אמרנו לו שיכפלם פי שנים ועלו ס‫' |
|- | |- | ||
| | | | ||
| − | + | ::We tell him to divide it in half; the result is 30. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואמרנו לו שיחלקם לחצי ועלו ל‫' |
|- | |- | ||
| | | | ||
| − | + | ::We have another half: we tell him to duplicate this half twice; the result is 90. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|והנה יש לנו חצי עו' אמרנו לו שיכפול זה החצי שהוא ל' פי שנים ועלו צ‫' |
|- | |- | ||
| | | | ||
| − | + | ::We tell him to cast out the nines; he has nothing left. | |
| − | |style="text-align:right;"|ט | + | |style="text-align:right;"|עו' אמרנו לו שישליכם ט'ט' והנה לא ישאר בידו כלום |
|- | |- | ||
| | | | ||
| − | + | ::So, there is 10 times 9 there. Take 2 for every 9; the result is 20, as the 20 he thought of. | |
| − | |style="text-align:right;"|י | + | |style="text-align:right;"|הרי שיש שם י' פעמים ט' ובעבו' כל ט' תקח ב' ועלו כ' כמו שהוא חשב כ‫' |
|- | |- | ||
| − | | | + | | colspan="2"| |
| − | + | ::<math>\scriptstyle{\color{blue}{\frac{\left[2\sdot\frac{\left(2\sdot20\right)+20}{2}\right]+\frac{\left(2\sdot20\right)+20}{2}}{9}\sdot2=\frac{\left(2\sdot\frac{60}{2}\right)+\frac{60}{2}}{9}\sdot2=\frac{\left(2\sdot30\right)+30}{9}\sdot2=\frac{90}{9}\sdot2=10\sdot2=20}}</math> | |
| − | |||
|- | |- | ||
| | | | ||
| − | * | + | :*Example, when he has more than 9 left: as if he thought of 9. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|דמיון לכשישאר בידו יותר מט' כגון שחשב ט‫' |
|- | |- | ||
| | | | ||
| − | + | ::We tell him to duplicate it twice; the result is 27. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|אמרנו לו שיכפלם פי שנים ועלו כ"ז |
|- | |- | ||
| | | | ||
| − | + | ::We tell him to divide it in half; the result is 13 and a half. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואמרנו לו שיחלקם לחצי ועלו י"ג וחצי |
|- | |- | ||
| | | | ||
| − | + | ::Since there is a half there; we tell him to think of it as a whole number; it is 14. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ולפי שיש כן חצי אמרנו שיחשבהו שלם ויהיו י"ד |
|- | |- | ||
| | | | ||
| − | + | ::We tell him to duplicate the 14 twice; the result is 42. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|עו' אמרנו לו שיכפול אלו הי"ד פי שנים ועלו מ"ב |
|- | |- | ||
| | | | ||
| − | + | ::We tell him to cast out the nines; he has 6 left. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|עו' אמרנו לו שישליכם ט'ט' ונשארו בידו ו‫' |
|- | |- | ||
| | | | ||
| − | + | ::So, there is 4 times 9 there. Take 2 for every 9; it is 8. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|הרי שיש בהם ד' פעמים ט' ובעבו' כל ט' תקח ב' הרי ח‫' |
|- | |- | ||
| | | | ||
| − | + | ::Take 1 for the remainder; it is 9, as the 9 he thought of. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ובעבו' הנשארי' תקח א' הרי ט' כמו ט' שחשב הוא |
|- | |- | ||
| − | | | + | | colspan="2"| |
| − | + | ::<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle\frac{\left[2\sdot\frac{\left(2\sdot9\right)+9}{2}\right]+\frac{\left(2\sdot9\right)+9}{2}}{9}\sdot2&\scriptstyle=\frac{\left(2\sdot\frac{27}{2}\right)+\frac{27}{2}}{9}\sdot2=\frac{\left[2\sdot\left(13+\frac{1}{2}\right)\right]+\left(13+\frac{1}{2}\right)}{9}\sdot2\\&\scriptstyle\approx\frac{\left(2\sdot14\right)+14}{9}\sdot2=\frac{42}{9}\sdot2=\left(4+\frac{6}{9}\right)\sdot2\\&\scriptstyle\approx\left(4\sdot2\right)+1=8+1=9\\\end{align}}}</math> | |
| − | |||
|- | |- | ||
| | | | ||
| − | * | + | *{{#annot:chosen number|667|nr3t}}To know [the number] a man thinks of, tell him to double it, add 5 [to the result], and multiply [the sum] by 5. |
| − | |style="text-align:right;"| | + | :Then he should add 10 [to the product], multiply [the sum] by 10, and subtract 350 from [the product]. |
| + | :Ask him what remained and take its analogous, i.e.: | ||
| + | :*if 100 remained, its analogous is 1, so say that he thought of 1; | ||
| + | :*if 200 remained, its analogous is 2, so say that he thought of 2; | ||
| + | :*if 300 remained, its analogous is 3, so say that he thought of 3; | ||
| + | :*and so on. | ||
| + | :<math>\scriptstyle\frac{\left[10\sdot\left[\left[5\sdot\left[\left(2\sdot x\right)+5\right]\right]+10\right]\right]-350}{100}=x</math> | ||
| + | |style="text-align:right;"|‫<ref>MS Ithaca: this problem appears at the end of the treatise, 46v</ref><big>לדעת מה שיחשוב האדם</big>‫<ref>MS Verona אחר</ref> אמור לו שיחשוב ויכפלם ויוסיף עליהם ה' וירבה אותם על ה‫'<br> | ||
| + | ויוסיף עליהם י' וירבה אותם על י' וישליך מהם ש"נ<br> | ||
| + | ושאל הנשאר ותקח דמיונם<br> | ||
| + | דהיינו שאם נשארו ק' שדמיונם א' אמור כי חשב א‫'<br> | ||
| + | ואם נשארו ר'‫<ref>ר': MS Ithaca om.</ref> שדמיונם ב' אמור כי חשב ב‫'<br> | ||
| + | ואם נשארו ש' שדמיונם ג' אמור כי חשב ג‫'<br> | ||
| + | וכן לעולם{{#annotend:nr3t}}‫<ref>MS Ithaca end.</ref> | ||
|- | |- | ||
| | | | ||
| − | * | + | :*Example: if he thought of 10. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|דמיון זה כגון שחשב י‫' |
|- | |- | ||
| | | | ||
| − | + | ::We tell him to duplicate it; the result is 20. | |
| − | |style="text-align:right;"|כ | + | |style="text-align:right;"|אמרנו לו שיכפלם ויהיו כ‫' |
|- | |- | ||
| | | | ||
| − | + | ::We tell him to add 5 to it; it is 25. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|גם אמרנו לו שיוסיף עליהם ה' ויהיו כ"ה |
|- | |- | ||
| | | | ||
| − | + | ::We tell him to multiply it by 5; it is 125. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|גם אמרנו לו שירבה אותם על ה' ויהיו קכ"ה |
|- | |- | ||
| | | | ||
| − | + | ::We tell him to add 10 to it; it is 135. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|עו' אמרנו לו שיוסיף עליהם י' ויהיו קל"ה |
|- | |- | ||
| | | | ||
| − | + | ::We tell him to multiply it by 10; it is one thousand and 350. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|גם אמרנו לו שירבה אותם על י' ויהיו אלף וש"נ |
|- | |- | ||
| | | | ||
| − | + | ::Subtract 350 from it; one thousand remains. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|השלך מהם ש"נ ישארו אלף |
|- | |- | ||
| | | | ||
| − | + | ::We take its analogous; it is 10, as [the number] he thought of. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ונקח דמיונם והוא י' כמו שחשב |
| + | |- | ||
| + | | colspan="2"| | ||
| + | ::<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle\frac{\left[10\sdot\left[\left[5\sdot\left[\left(2\sdot10\right)+5\right]\right]+10\right]\right]-350}{100}&\scriptstyle=\frac{\left[10\sdot\left[\left[5\sdot\left(20+5\right)\right]+10\right]\right]-350}{100}\\&\scriptstyle=\frac{\left[10\sdot\left[\left(5\sdot25\right)+10\right]\right]-350}{100}\\&\scriptstyle=\frac{\left[10\sdot\left(125+10\right)\right]-350}{100}\\&\scriptstyle=\frac{\left(10\sdot135\right)-350}{100}\\&\scriptstyle=\frac{1350-350}{100}=\frac{1000}{100}=10\\\end{align}}}</math> | ||
|- | |- | ||
| | | | ||
| − | * | + | *{{#annot:chosen number|667|wl7G}}Tell him to multiply [the number] he thought of by 3, multiply [the result] by 4, multiply [the product] by 5, then divide [the result] by [the number] he thought of at first. |
| − | |style="text-align:right;"| | + | :You can guess the result by applying this calculation on 1. |
| + | :<math>\scriptstyle\frac{x\sdot3\sdot4\sdot5}{x}=3\sdot4\sdot5</math> | ||
| + | |style="text-align:right;"|אחר אמור שיחשוב ויכפול מה שחשב על ג' כן יכפלהו על ד' א"כ יכפלהו על ה' ויחלקהו על מה שחשב בראשונה<br> | ||
| + | ותוכל לדעת הנשאר בעשותך החשבון הזה על א‫'{{#annotend:wl7G}} | ||
|- | |- | ||
| | | | ||
| − | * | + | :*Example: if he thought of the number 4 |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|דמיון זה כגון שחשב ד‫' |
|- | |- | ||
| | | | ||
| − | + | ::Multiply it by 3; it is 12. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כפול אותם על ג' ויהיו י"ב |
|- | |- | ||
| | | | ||
| − | + | ::Multiply it by 4; it is 48. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כפול אותם על ד' ויהיו מ"ח |
|- | |- | ||
| | | | ||
| − | + | ::Multiply it by 5; it is 240. | |
| − | |style="text-align:right;"|מ | + | |style="text-align:right;"|כפול אותם על ה' ויהיו ר"מ |
|- | |- | ||
| | | | ||
| − | + | ::Divide it by the 4 he originally thought of; he is left with 60. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|חלקם על ד' שחשב בראשונה נשארו בידו ס‫' |
| + | |- | ||
| + | | colspan="2"| | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{4\sdot3\sdot4\sdot5}{4}=\frac{12\sdot4\sdot5}{4}=\frac{48\sdot5}{4}=\frac{240}{4}=60}}</math> | ||
|- | |- | ||
| | | | ||
| − | + | ::Whereas you thing of 1. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ואתה חשבת א‫' |
|- | |- | ||
| | | | ||
| − | + | ::You multiply it by 3; it is 3. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כפלת אותו על ג' היו ג‫' |
|- | |- | ||
| | | | ||
| − | + | ::You multiply it by 4; it is 12. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כפלת אותו על ד' יהיו י"ב |
|- | |- | ||
| | | | ||
| − | + | ::You multiply it by 5; it is 60. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|כפלת אותו על ה' היו ס‫' |
|- | |- | ||
| | | | ||
| − | + | ::You divide it by 1; it is 60, as he has left. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|חלקת אותו על א' יהיו ס' כמו שנשאר בידו |
| + | |- | ||
| + | | colspan="2"| | ||
| + | ::<math>\scriptstyle{\color{blue}{\frac{1\sdot3\sdot4\sdot5}{1}=\frac{3\sdot4\sdot5}{1}=\frac{12\sdot5}{1}=\frac{60}{1}=60}}</math> | ||
|- | |- | ||
| | | | ||
| − | * | + | *{{#annot:chosen number|667|82ev}}Tell him to think of any number that he wishes, but not less than 4, add twice of it, and halve the result. |
| − | |style="text-align:right;"| | + | :If there is a fraction in half the result, he should consider it as a whole; if not, there is no need for another correction. |
| + | :Then he should take the half, add twice of it, and halve the result. | ||
| + | :If there is a fraction in half the result, he should consider it as a whole, as before. | ||
| + | :Tell him to divide the result by 9 and let you know the number resulted from the division. | ||
| + | :As he tells you the number, multiply it by 4. | ||
| + | :Now you should make the result more accurate: when you asked him twice if there was or was not a fraction in half the result - | ||
| + | :*If he answered on the first time [there are] fractions [in half the result], and on the second time wholes [only], add 1 to your result. | ||
| + | :*If on the first time he answered wholes [only] and on the second time [there were] fractions, add 2 to your result. | ||
| + | :*If he answered on both the first and the second times [that there are] fractions [in half the result] add 3 to your result. | ||
| + | :*If he answered on both the first and the second times [that there are] wholes [in half the result] there is no need for another correction - what he thought of is what you get when multiplying his result of division by 4. | ||
| + | :<math>\scriptstyle x\ge4\longrightarrow\frac{\frac{\left(2\sdot\frac{2x+x}{2}\right)+\frac{2x+x}{2}}{2}}{9}\sdot4=x</math> | ||
| + | |style="text-align:right;"|אחר אמור שיחשוב אי זה מספר שירצה אך לא יהיה פחות מד' ויוסיף עליו פי שנים ויחלק העולה לחצאים<br> | ||
| + | אם יש בחצי שבר יחשבהו שלם ואם לאו אין צריך תקון אחר<br> | ||
| + | ויקח החצי ויוסיף עליו פי שנים ויחלק העולה לחצאים<br> | ||
| + | ואם יש בחצי שבר יחשבהו שלם כאשר בתחלה<br> | ||
| + | ואמור לו שיחלק מה שיעלה בידו על ט' ויגיד לך מספר מה שיעלה בחלוק<br> | ||
| + | וכאשר הגיד לך המספר תקח לך בעבו' כל אחד ממספר שעלה בחלוק ד‫'<br> | ||
| + | וכאשר עלה אמנם צריך אתה לדקדק עו‫'<br> | ||
| + | כי אם כאשר שאלת לו פעמים אם יש בחצי שבר או לאו והשיב לך בפעם הראשונה שבורי' ובשנייה שלמים תוסיף על מה שעלה בידיך א‫'<br> | ||
| + | ואם השיב בראשונה שלמים ובשנייה שבורי' תוסיף על מה שעלה בידיך ב‫'<br> | ||
| + | ואם השיב בראשונה ובשנייה שבורים תוסיף על מה שעלה בידיך ג‫'<br> | ||
| + | ואם השיב בראשונה ובשנייה שלמים אינך צריך עוד התקון אחר רק כאשר עולה בידיך כשלקח ד' לכל אחד ממה שעשה בחלוק כמה שחשב והבן זה{{#annotend:82ev}} | ||
| + | |- | ||
| + | |<span style=color:green>'''endnote - MS Verona:'''</span> These methods have been completed, blessed be He, Who dwells on the highest heights, Amen, may the writer be strong and the reader be courageous. | ||
| + | |style="text-align:right;"|תמו אלו הדרכים ברוך שוכן עליונים אמן חזק הכותב ואמץ הקורא | ||
|- | |- | ||
| + | |<span style=color:green>additional problem - another version of a guessing problem discussed above</span> | ||
| | | | ||
| − | |||
| − | |||
|- | |- | ||
| | | | ||
| − | * | + | *{{#annot:chosen number|667|q6LW}}Tell you friend to choose a number |
| − | |style="text-align:right;"| | + | :Then tell him to cast out the threes from it, and tell you the remainder - for each one that remained [take] 70. |
| + | :*[The sign for this is] 3, 70. | ||
| + | :Then tell him to cast out the fives from the number he thought of, and tell you the remainder - for each one that remained [take] 21. | ||
| + | :*The sign for this is 5, 21. | ||
| + | :Afterwards tell him to cast out the sevens from the number he chose, and tell you the remainder - for each one that remained [take] 15. | ||
| + | :*The sign for this is 100, 15. | ||
| + | :Then sum up [the results] and cast out 105 repeatedly. | ||
| + | :The remainder will be the number he chose. | ||
| + | |style="text-align:right;"|ג"ע הכ"א קט"ו<br> | ||
| + | כשתרצה לעשות זה החשבון אמור לחברך שיחשוב אי זה חשבון שתרצה<br> | ||
| + | ואחר שחשב תאמ' לו להשליכם ג' ג' והוא יאמ' לך הנשאר וכל אחד אשר ישאר ע' הוא ע‫'<br> | ||
| + | ג"ע<br> | ||
| + | א"כ תאמ' לו השלך מה שחשבת ה' ה' והוא יאמ' לך הנשאר וכל אחד שתאמר עתה הוא כ"א<br> | ||
| + | וזה סימן הכ"א<br> | ||
| + | א"כ אמר לו השלך מה שחשבת ז' ז' והוא יאמ' לך הנשאר וכל אחד שישאר עתה הוא ט"ו<br> | ||
| + | וזה סימן קט"ו<br> | ||
| + | א"כ כלול הנשאר יחד והוציא מהמספר ק"ה ומה שישאר בידך הוא המספר שחשב חברך{{#annotend:q6LW}} | ||
| + | |- | ||
| + | | colspan="2"| | ||
| + | :<math>\scriptstyle\left[\left[\left(x\, mod3\right)\sdot70\right]+\left[\left(x\, mod5\right)\sdot21\right]+\left[\left(x\, mod7\right)\sdot15\right]\right]mod105=x</math> | ||
|- | |- | ||
| | | | ||
| − | * | + | :*Example: if your friend thought of [the number] 11. |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|דמיון זה הרי שחשב חברך י"א |
|- | |- | ||
| | | | ||
| − | + | ::Cast out the threes; 2 remains. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|השליכם ג' ג' ישאר ב‫' |
|- | |- | ||
| | | | ||
| − | + | ::Cast out the fives; 1 remains. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|השליכם [ה' ה'] ישאר א‫' |
|- | |- | ||
| | | | ||
| − | + | ::Cast out the sevens; 4 remains. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|השליכם ז' ז' ישאר [ד‫'] |
|- | |- | ||
| | | | ||
| − | + | ::Sum up [...] | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|[...] כלול הכל |
|- | |- | ||
| | | | ||
| − | + | ::[...] two times 70; it is 140. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|שתי פעמי' ע' הם ק"מ |
|- | |- | ||
| | | | ||
| − | + | ::One time 21; it is 21. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|א' פעם כ"א הוא כ"א |
|- | |- | ||
| | | | ||
| − | + | ::4 times 15; it is 60. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ד' פעמי' ט"ו הם ס‫' |
|- | |- | ||
| | | | ||
| − | + | ::Sum up 140, 21 and 60; the result is 221. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|חבר ק"מ וכ"א וס' יעלו רכ"א |
|- | |- | ||
| | | | ||
| − | + | ::Cast out 105 twice; 11 remains, which is as [the number] your friend thought of. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|השלך מהם שתי פעמים ק"ה ישאר י"א כמו שחשב חברך |
| + | |- | ||
| + | | colspan="2"| | ||
| + | ::<math>\scriptstyle{\color{blue}{\begin{align}\scriptstyle x&\scriptstyle=\left[\left[\left(11\, mod3\right)\sdot70\right]+\left[\left(11\, mod5\right)\sdot21\right]+\left[\left(11\, mod7\right)\sdot15\right]\right]mod105\\&\scriptstyle=\left[\left(2\sdot70\right)+\left(1\sdot21\right)+\left(4\sdot15\right)\right]mod105\\&\scriptstyle=\left(140+21+60\right)mod105\\&\scriptstyle=221mod105=221-\left(2\sdot105\right)=11\\\end{align}}}</math> | ||
|- | |- | ||
| | | | ||
| − | + | ::Know that if [the sum is] three [=should be four] hundreds or more - subtract from it 3 times 105. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|ודע שאם שלש מאות או יותר תשליך מהם ג' פעמי' ק"ה |
|- | |- | ||
| | | | ||
| − | + | ::[If the sum is] five [hundreds] - [subtract from it] 4 times 105 | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכן לה' ד' פעמי' ק"ה |
|- | |- | ||
| | | | ||
| − | + | ::And so on. | |
| − | |style="text-align:right;"| | + | |style="text-align:right;"|וכן כלם |
|- | |- | ||
| | | | ||
| − | *63 - divisible by 3, 7, 9, (21 is missing) | + | |
| − | |style="text-align:right;"|ס"ג יש לו שליש ושביע ותשיע | + | === <span style=color:green>Divisibility of the Numbers 1-112 (MS Ithaca)</span> === |
| − | |- | + | |
| − | | | + | | |
| − | *64 - divisible by 2, 4, 8, 16, 32 | + | |- |
| − | |style="text-align:right;"|ס"ד יש לו חצי ורביע ושמיני וי"ו ול"ב | + | |<span style=color:green>'''endnote - MS Ithaca:'''</span> The arithmetic book is complete. |
| − | |- | + | |style="text-align:right;"|<big>נשלם ספר החשבון</big> |
| − | | | + | |- |
| − | *65 - divisible by 5, 13 | + | |Praise be to God, who teaches wisdom to every wise and intelligent person. |
| − | |style="text-align:right;"|ס"ה יש לו חמש וי"ג | + | |style="text-align:right;"|<big>תהלה לאל המחכים לכל חכם ונבון</big> |
| − | |- | + | |- |
| − | | | + | |Completed and Finished. |
| − | *66 - divisible by 2, 3, 6, 11, 22, 33 | + | |style="text-align:right;"|תם ונשלם |
| − | |style="text-align:right;"|ס"ו יש לו חצי ושליש ושתות וי"א וכ"ב ול"ג | + | |- |
| − | |- | + | |These are the numbers that have [divisors], i.e. they are divided into halves, thirds, quarters and their like and those that do not. |
| − | | | + | |style="text-align:right;"|‫<ref>46r</ref>אלו הם חשבונות שיש להם ריגולא ר"ל שיש להם חלוק לחצי ולשליש ולרביע ולדומים ואותן שאין להם ריגולא |
| − | + | |- | |
| − | |style="text-align:right;"|ס"ז אין לו ריגולא | + | |1 has no [divisor]. |
| − | |- | + | |style="text-align:right;"|א' אין לו ריגולא |
| − | | | + | |- |
| − | *68 - divisible by 2, 4, 17, 34 | + | |2 has a [divisor], because it has a half. |
| − | |style="text-align:right;"|ס"ח יש לו חצי ורביע וי"ז ול"ד | + | |style="text-align:right;"|ב' יש לו ריגולא כי יש לו חצי |
| − | |- | + | |- |
| − | | | + | |3 has a third. |
| − | *69 - divisible by 3, 23 | + | |style="text-align:right;"|ג' יש לו שליש |
| − | |style="text-align:right;"|ס"ט יש לו שליש וכ"ג | + | |- |
| − | |- | + | |4 has a half and a quarter. |
| − | | | + | |style="text-align:right;"|ד' יש לו חצי ורביע |
| − | *70 - divisible by 2, 7, 5, 14, 35, (10 is missing) | + | |- |
| − | |style="text-align:right;"|ע' יש לו חצי ושביע וחמש וי"ד ול"ה | + | |5 has no [divisor]. |
| − | |- | + | |style="text-align:right;"|ה' אין לו ריגולא |
| − | | | + | |- |
| − | + | |6 has a half and a third, and it is equal to [the sum] of its fractions [= a perfect number]. | |
| − | |style="text-align:right;"|ע"א אין לו ריגולא | + | :<math>\scriptstyle{\color{OliveGreen}{6=\left(\frac{1}{2}\sdot6\right)+\left(\frac{1}{3}\sdot6\right)+\left(\frac{1}{6}\sdot6\right)}}</math> |
| − | |- | + | |style="text-align:right;"|ו' יש לו חצי ושליש והוא שוה בחלקיו |
| − | | | + | |- |
| − | *72 - divisible by 2, 3, 4, 6, 8, 9, 12, 18, 24, 36 | + | |7 has no [divisor]. |
| − | |style="text-align:right;"|ע"ב יש לו חצי ושליש ורביע ושתות ושמיני ותשיע י"ב י"ח כ"ד ל"ו | + | |style="text-align:right;"|ז' אין לו ריגולא |
| − | |- | + | |- |
| − | | | + | |8 has a half and a quarter. |
| − | + | |style="text-align:right;"|ח' יש לו חצי ורביע | |
| + | |- | ||
| + | |9 has a third. | ||
| + | |style="text-align:right;"|ט' יש לו שליש | ||
| + | |- | ||
| + | |10 has a half and a fifth. | ||
| + | |style="text-align:right;"|י' יש לו חצי וחמש | ||
| + | |- | ||
| + | |11 has no [divisor]. | ||
| + | |style="text-align:right;"|י"א אין לו ריגולא | ||
| + | |- | ||
| + | |12 has a half, a third, a quarter and a sixth. | ||
| + | |style="text-align:right;"|י"ב יש לו חצי ושליש ורביע ושתות | ||
| + | |- | ||
| + | |13 has no [divisor]. | ||
| + | |style="text-align:right;"|י"ג אין לו ריגולא | ||
| + | |- | ||
| + | |14 has a half and a seventh. | ||
| + | |style="text-align:right;"|י"ד יש לו חצי ושביע | ||
| + | |- | ||
| + | |15 has a third and a fifth. | ||
| + | |style="text-align:right;"|ט"ו יש לו שליש וחמש | ||
| + | |- | ||
| + | |16 has a half, a quarter and an eighth. | ||
| + | |style="text-align:right;"|י"ו יש לו חצי ורביע ושמיני | ||
| + | |- | ||
| + | |17 has no [divisor]. | ||
| + | |style="text-align:right;"|י"ז אין לו ריגולא | ||
| + | |- | ||
| + | |18 has a half, a third, a sixth [and a ninth]. | ||
| + | |style="text-align:right;"|י"ח יש לו חצי ושליש ושתות | ||
| + | |- | ||
| + | |19 has no [divisor]. | ||
| + | |style="text-align:right;"|י"ט אין לו ריגולא | ||
| + | |- | ||
| + | |20 has a half, a quarter, a fifth [and a tenth]. | ||
| + | |style="text-align:right;"|כ' יש לו חצי ורביע וחמש | ||
| + | |- | ||
| + | |21 has a third and a seventh. | ||
| + | |style="text-align:right;"|כ"א יש לו שליש ושביע | ||
| + | |- | ||
| + | |22 has a half and one [part] of 11. | ||
| + | |style="text-align:right;"|כ"ב יש לו חצי ואחד מי"א | ||
| + | |- | ||
| + | |23 has no [divisor]. | ||
| + | |style="text-align:right;"|כ"ג אין לו ריגולא | ||
| + | |- | ||
| + | |24 has a half, a third, a quarter, a sixth, an eighth [and one part of 12]. | ||
| + | |style="text-align:right;"|כ"ד יש לו חצי ושליש ורביע ושתות [ו]שמיני | ||
| + | |- | ||
| + | |25 has a fifth. | ||
| + | |style="text-align:right;"|כ"ה יש לו חמש | ||
| + | |- | ||
| + | |26 has a half and one [part] of 13. | ||
| + | |style="text-align:right;"|כ"ו יש לו חצי ואחד מי"ג | ||
| + | |- | ||
| + | |27 has a third and a ninth. | ||
| + | |style="text-align:right;"|כ"ז יש לו שליש ותשיע | ||
| + | |- | ||
| + | |28 has a half, a quarter, a seventh and one [part] of 14. | ||
| + | |style="text-align:right;"|כ"ח יש לו חצי ורביע ושביע ואחד מי"ד | ||
| + | |- | ||
| + | |29 has no [divisor]. | ||
| + | |style="text-align:right;"|כ"ט אין לו רגולא | ||
| + | |- | ||
| + | |30 has a half, a third, a tenth, one [part] of 15, [a fifth and a sixth] | ||
| + | |style="text-align:right;"|ל' יש לו חצי ושליש ועשירית ואחד מט"ו | ||
| + | |- | ||
| + | |31 has no [divisor]. | ||
| + | |style="text-align:right;"|ל"א אין לו ריגולא | ||
| + | |- | ||
| + | |32 has a half, a quarter, an eighth, and one [part] of 16. | ||
| + | |style="text-align:right;"|ל"ב יש לו חצי ורביעי' ושמינית ואחד מי"ו | ||
| + | |- | ||
| + | |33 has a third and one [part] of 11. | ||
| + | |style="text-align:right;"|ל"ג יש לו שליש ואחד מי"א | ||
| + | |- | ||
| + | |34 has a half and one [part] of 17. | ||
| + | |style="text-align:right;"|ל"ד יש לו חצי ואחד מי"ז | ||
| + | |- | ||
| + | |35 has a fifth and a seventh. | ||
| + | |style="text-align:right;"|ל"ה יש לו חמש ושביע | ||
| + | |- | ||
| + | |36 has a half, a third, a ninth, a quarter [one part of 12, and one part of 18]. | ||
| + | |style="text-align:right;"|ל"ו יש לו חצי ושליש ותשיע ורביע | ||
| + | |- | ||
| + | |37 has no [divisor]. | ||
| + | |style="text-align:right;"|ל"ז אין לו ריגולא | ||
| + | |- | ||
| + | |38 has a half and one [part] of 19. | ||
| + | |style="text-align:right;"|ל"ח יש לו חצי ואחד מי"ט | ||
| + | |- | ||
| + | |39 has a third and one [part] of 13. | ||
| + | |style="text-align:right;"|ל"ט יש לו שליש ואחד מי"ג | ||
| + | |- | ||
| + | |40 has a half, a quarter, a fifth, an eighth, a tenth [and one part of 20]. | ||
| + | |style="text-align:right;"|מ' יש לו חצי ורביע וחמש ושמין ועשירי | ||
| + | |- | ||
| + | |41 has no [divisor]. | ||
| + | |style="text-align:right;"|מ"א אין לו ריגולא | ||
| + | |- | ||
| + | | | ||
| + | *42 - divisible by 2, 3, 6, 7, 14, 21 | ||
| + | |style="text-align:right;"|מ"ב יש לו חצי ושליש ושתות ושביע וי"ד וכ"א | ||
| + | |- | ||
| + | |43 has no [divisor]. | ||
| + | |style="text-align:right;"|מ"ג אין לו ריגולא | ||
| + | |- | ||
| + | | | ||
| + | *44 - divisible by 2, 4, 11, 22 | ||
| + | |style="text-align:right;"|מ"ד יש לו חצי ורביע וי"א וכ"ב | ||
| + | |- | ||
| + | | | ||
| + | *45 - divisible by 5, 9, 15, (3 is missing) | ||
| + | |style="text-align:right;"|מ"ה יש לו חמש ותשיע וט"ו | ||
| + | |- | ||
| + | | | ||
| + | *46 - divisible by 2, 23 | ||
| + | |style="text-align:right;"|מ"ו יש לו חצי ואחד מכ"ג | ||
| + | |- | ||
| + | |47 has no [divisor]. | ||
| + | |style="text-align:right;"|מ"ז אין לו ריגולא | ||
| + | |- | ||
| + | | | ||
| + | *48 - divisible by 2, 3, 4, 6, 8, 12, 16, 24 | ||
| + | |style="text-align:right;"|מ"ח יש לו חצי ושליש ורביע ושתות ושמין י"ב וי"ו וכ"ד | ||
| + | |- | ||
| + | | | ||
| + | *49 - divisible by 7 | ||
| + | |style="text-align:right;"|מ"ט יש לו שביע | ||
| + | |- | ||
| + | | | ||
| + | *50 - divisible by 2, 5, 10, 25 | ||
| + | |style="text-align:right;"|נ' יש לו חצי וחמש ועשירית וכ"ה | ||
| + | |- | ||
| + | | | ||
| + | *51 - divisible by 3, 17 | ||
| + | |style="text-align:right;"|נ"א יש לו שליש וי"ז | ||
| + | |- | ||
| + | | | ||
| + | *52 - divisible by 2, 4, 13, 26 | ||
| + | |style="text-align:right;"|נ"ב יש לו חצי ורביע וי"ג וכ"ו | ||
| + | |- | ||
| + | |53 has no [divisor]. | ||
| + | |style="text-align:right;"|נ"ג אין לו ריגולא | ||
| + | |- | ||
| + | | | ||
| + | *54 - divisible by 2, 3, 6, 9, 27, (18 is missing) | ||
| + | |style="text-align:right;"|נ"ד יש לו חצי ושליש ושתות ותשיע וכ"ז | ||
| + | |- | ||
| + | | | ||
| + | *55 - divisible by 5, 11 | ||
| + | |style="text-align:right;"|נ"ה יש לו חמש וי"א | ||
| + | |- | ||
| + | | | ||
| + | *56 - divisible by 2, 4, 7, 8, 14, 28 | ||
| + | |style="text-align:right;"|נ"ו יש לו חצי ורביע ושביע ושמיני וי"ד וכ"ח | ||
| + | |- | ||
| + | | | ||
| + | *57 - divisible by 3, 19 | ||
| + | |style="text-align:right;"|נ"ז יש לו שליש וי"ט | ||
| + | |- | ||
| + | | | ||
| + | *58 - divisible by 2, 29 | ||
| + | |style="text-align:right;"|נ"ח יש לו חצי וכ"ט | ||
| + | |- | ||
| + | |59 has no [divisor]. | ||
| + | |style="text-align:right;"|נ"ט אין לו ריגולא | ||
| + | |- | ||
| + | | | ||
| + | *60 - divisible by 2, 3, 4, 5, 6, 10, 12, 15, 20, 30 | ||
| + | |style="text-align:right;"|‫<ref>46v</ref>ס' יש לו חצי ושליש ורביע וחמש ושתות ועשירי וי"ב וט"ו וכ' ול‫' | ||
| + | |- | ||
| + | |61 has no [divisor]. | ||
| + | |style="text-align:right;"|ס"א אין לו ריגולא | ||
| + | |- | ||
| + | | | ||
| + | *62 - divisible by 2, 31 | ||
| + | |style="text-align:right;"|ס"ב יש לו חצי ול"א | ||
| + | |- | ||
| + | | | ||
| + | *63 - divisible by 3, 7, 9, (21 is missing) | ||
| + | |style="text-align:right;"|ס"ג יש לו שליש ושביע ותשיע | ||
| + | |- | ||
| + | | | ||
| + | *64 - divisible by 2, 4, 8, 16, 32 | ||
| + | |style="text-align:right;"|ס"ד יש לו חצי ורביע ושמיני וי"ו ול"ב | ||
| + | |- | ||
| + | | | ||
| + | *65 - divisible by 5, 13 | ||
| + | |style="text-align:right;"|ס"ה יש לו חמש וי"ג | ||
| + | |- | ||
| + | | | ||
| + | *66 - divisible by 2, 3, 6, 11, 22, 33 | ||
| + | |style="text-align:right;"|ס"ו יש לו חצי ושליש ושתות וי"א וכ"ב ול"ג | ||
| + | |- | ||
| + | |67 has no [divisor]. | ||
| + | |style="text-align:right;"|ס"ז אין לו ריגולא | ||
| + | |- | ||
| + | | | ||
| + | *68 - divisible by 2, 4, 17, 34 | ||
| + | |style="text-align:right;"|ס"ח יש לו חצי ורביע וי"ז ול"ד | ||
| + | |- | ||
| + | | | ||
| + | *69 - divisible by 3, 23 | ||
| + | |style="text-align:right;"|ס"ט יש לו שליש וכ"ג | ||
| + | |- | ||
| + | | | ||
| + | *70 - divisible by 2, 7, 5, 14, 35, (10 is missing) | ||
| + | |style="text-align:right;"|ע' יש לו חצי ושביע וחמש וי"ד ול"ה | ||
| + | |- | ||
| + | |71 has no [divisor]. | ||
| + | |style="text-align:right;"|ע"א אין לו ריגולא | ||
| + | |- | ||
| + | | | ||
| + | *72 - divisible by 2, 3, 4, 6, 8, 9, 12, 18, 24, 36 | ||
| + | |style="text-align:right;"|ע"ב יש לו חצי ושליש ורביע ושתות ושמיני ותשיע י"ב י"ח כ"ד ל"ו | ||
| + | |- | ||
| + | |73 has no [divisor]. | ||
|style="text-align:right;"|ע"ג אין לו ריגולא | |style="text-align:right;"|ע"ג אין לו ריגולא | ||
|- | |- | ||
| Line 6,524: | Line 8,384: | ||
|style="text-align:right;"|ע"ח יש לו חצי ושתות וי"ג ול"ט | |style="text-align:right;"|ע"ח יש לו חצי ושתות וי"ג ול"ט | ||
|- | |- | ||
| − | | | + | |79 has no [divisor]. |
| − | |||
|style="text-align:right;"|ע"ט אין לו ריגולא | |style="text-align:right;"|ע"ט אין לו ריגולא | ||
|- | |- | ||
| Line 6,540: | Line 8,399: | ||
|style="text-align:right;"|פ"ב יש לו חצי ומ"א | |style="text-align:right;"|פ"ב יש לו חצי ומ"א | ||
|- | |- | ||
| − | | | + | |83 has no [divisor]. |
| − | |||
|style="text-align:right;"|פ"ג אין לו ריגולא | |style="text-align:right;"|פ"ג אין לו ריגולא | ||
|- | |- | ||
| Line 6,564: | Line 8,422: | ||
|style="text-align:right;"|פ"ח יש לו חצי וי"א ושמיני ורביע וכ"ב ומ"ד | |style="text-align:right;"|פ"ח יש לו חצי וי"א ושמיני ורביע וכ"ב ומ"ד | ||
|- | |- | ||
| − | | | + | |89 has no [divisor]. |
| − | |||
|style="text-align:right;"|פ"ט אין לו ריגולא | |style="text-align:right;"|פ"ט אין לו ריגולא | ||
|- | |- | ||
| Line 6,596: | Line 8,453: | ||
|style="text-align:right;"|צ"ו יש לו חצי ושליש ורביע ושתות ושמיני וי"ב וי"ו וכ"ד ול"ב ומ"ח | |style="text-align:right;"|צ"ו יש לו חצי ושליש ורביע ושתות ושמיני וי"ב וי"ו וכ"ד ול"ב ומ"ח | ||
|- | |- | ||
| − | | | + | |97 has no [divisor]. |
| − | |||
|style="text-align:right;"|צ"ז אין לו ריגולא | |style="text-align:right;"|צ"ז אין לו ריגולא | ||
|- | |- | ||
| Line 6,612: | Line 8,468: | ||
|style="text-align:right;"|ק' יש לו חצי ורביע וחמש ועשירי וכ' וכ"ה ונ‫' | |style="text-align:right;"|ק' יש לו חצי ורביע וחמש ועשירי וכ' וכ"ה ונ‫' | ||
|- | |- | ||
| − | | | + | |101 has no [divisor]. |
| − | |||
|style="text-align:right;"|ק"א אין לו ריגולא | |style="text-align:right;"|ק"א אין לו ריגולא | ||
|- | |- | ||
| Line 6,620: | Line 8,475: | ||
|style="text-align:right;"|ק"ב יש לו ריגולא חצי ושליש ושתות וי"ז ול"ד ונ"א | |style="text-align:right;"|ק"ב יש לו ריגולא חצי ושליש ושתות וי"ז ול"ד ונ"א | ||
|- | |- | ||
| − | | | + | |103 has no [divisor]. |
| − | |||
|style="text-align:right;"|ק"ג אין לו ריגולא | |style="text-align:right;"|ק"ג אין לו ריגולא | ||
|- | |- | ||
| Line 6,636: | Line 8,490: | ||
|style="text-align:right;"|ק"ו יש לו חצי ונ"ג | |style="text-align:right;"|ק"ו יש לו חצי ונ"ג | ||
|- | |- | ||
| − | | | + | |107 has no [divisor]. |
| − | |||
|style="text-align:right;"|ק"ז אין לו ריגולא | |style="text-align:right;"|ק"ז אין לו ריגולא | ||
|- | |- | ||
| Line 6,644: | Line 8,497: | ||
|style="text-align:right;"|ק"ח יש לו חצי ושליש ורביע ושתות ותשיע וי"ב וי"ח ול"ו ונ"ד | |style="text-align:right;"|ק"ח יש לו חצי ושליש ורביע ושתות ותשיע וי"ב וי"ח ול"ו ונ"ד | ||
|- | |- | ||
| − | | | + | |109 has no [divisor]. |
| − | |||
|style="text-align:right;"|ק"ט אין לו ריגולא | |style="text-align:right;"|ק"ט אין לו ריגולא | ||
|- | |- | ||
| Line 6,652: | Line 8,504: | ||
|style="text-align:right;"|ק"י יש לו חצי וחמש ועשירי וי"א וכ"ב ונ"ה | |style="text-align:right;"|ק"י יש לו חצי וחמש ועשירי וי"א וכ"ב ונ"ה | ||
|- | |- | ||
| − | | | + | |111 has no [divisor]. |
| − | |||
|style="text-align:right;"|קי"א אין לו ריגולא | |style="text-align:right;"|קי"א אין לו ריגולא | ||
|- | |- | ||
| Line 6,660: | Line 8,511: | ||
|style="text-align:right;"|קי"ב יש לו חצי ורביע וכ"ח ונ"ו | |style="text-align:right;"|קי"ב יש לו חצי ורביע וכ"ח ונ"ו | ||
|- | |- | ||
| − | | | + | |The end. |
|style="text-align:right;"|סליק | |style="text-align:right;"|סליק | ||
|- | |- | ||
| − | | | + | |Writings of Don Benveniste ben Labi. |
| − | |style="text-align:right;"|כתבים כ"ז, מהשר החכם דון בנבנשית בן לביא | + | |style="text-align:right;"|‫<ref>MS Ithaca end</ref>כתבים כ"ז, מהשר החכם דון בנבנשית בן לביא |
|- | |- | ||
|} | |} | ||
| − | == | + | == Apparatus == |
| + | |||
| + | <div style="text-align: right;"><div class="mw-collapsible mw-collapsed"><div class="mw-collapsible-content"> | ||
| + | <br> | ||
<references /> | <references /> | ||
| + | </div></div></div> | ||
== Appendix: Bibliography == | == Appendix: Bibliography == | ||
| Line 6,683: | Line 8,538: | ||
:[http://gallica.bnf.fr/ark:/12148/btv1b90645087/f35.image.r=Hébreu.langEN heb. 1049/2] | :[http://gallica.bnf.fr/ark:/12148/btv1b90645087/f35.image.r=Hébreu.langEN heb. 1049/2] | ||
:5) Verona, Biblioteca Civica 33 (83.1)/2 (IMHM: f 32674), ff. 8r-84v; 222r-v (Cento, 1461) | :5) Verona, Biblioteca Civica 33 (83.1)/2 (IMHM: f 32674), ff. 8r-84v; 222r-v (Cento, 1461) | ||
| + | |||
| + | <span style=color:blue>The transcript is based mainly on manuscript Ithaca 26</span> | ||
Latest revision as of 09:24, 29 November 2024
Contents
- 1 The Nature of One
- 2 Shortcuts - Products of Ranks by Ranks
- 3 The Decimal Ranks[61]
- 4 Three Types of Numbers[71]
- 5 The Ten Numerals[73]
- 6 The Method of Finding the Square Numbers[75]
- 7 Cubic Numbers[79]
- 8 Primary Definitions[80]
- 9 Multiplication
- 9.1 Units and tens by units and tens
- 9.2 Units by units and tens
- 9.3 Units, tens, and hundreds by units, tens, and hundreds
- 9.4 Units and tens by units, tens, and hundreds
- 9.5 Units by units, tens, and hundreds
- 9.6 Units, tens, hundreds, and thousands by units, tens, hundreds, and thousands
- 9.7 Units, tens, hundreds, and thousands by units, tens, and hundreds
- 9.8 Units, tens, hundreds, and thousands by units and tens
- 9.9 Units, tens, hundreds, and thousands by units
- 9.10 Units, tens, hundreds, thousands, and tens of thousands by units, tens, hundreds, thousands, and tens of thousands
- 9.11 Units, tens, hundreds, thousands, tens of thousands, and hundreds of thousands by units, tens, hundreds, thousands, tens of thousands, and hundreds of thousands
- 10 The Method of Summing Up Separate Numbers Called Congregare or Riunire
- 11 The Method of Subtracting a Number from a Number Called Sottrarre
- 12 The Method of Dividing a Number by a Number Called Dividere or Partire
- 13 The Method of Knowing the Numbers Summed One after the Other Successively
- 14 Word Problems - The Rule of Three
- 15 Calculation of Fractions
- 16 Word Problems
- 16.1 Exchange Problems
- 16.2 Divide a Quantity Problems
- 16.3 Partnership - for the same time
- 16.4 Multiple Quantities Problems
- 16.5 Joint Purchase Problem - If You Give Me - Amounts of Money
- 16.6 Multiple Quantities Problems
- 16.7 Mixture and Alligation Problem
- 16.8 Pricing Problems
- 16.9 Shared Work Problem - Four rivers
- 16.10 Multiple Quantities Problems
- 16.11 Rent Problems
- 16.12 Pricing Problems
- 16.13 Interest and Discount Problems - Find the Earned Interest
- 16.14 Interest and Discount Problems (MS Verona)
- 16.15 Find a Number Problems
- 16.16 Find the Price - Cubits of Cloth
- 16.17 Interest and Discount Problem - Compound Interest
- 16.18 Pricing Problems
- 16.19 Guessing Problems
- 16.20 Divide a Quantity Problem
- 16.21 Motion Problem - To and From
- 16.22 Find a Quantity Problem - First from Last
- 16.23 Ordering Problems
- 16.24 Find a Quantity Problem - Whole from Parts
- 16.25 Divide a Quantity Problem - Proportional Division
- 16.26 Buy and Sell Problem
- 16.27 Ordering Problems
- 16.28 Multiple Quantities Problems
- 16.29 Fractions of Fractions (MS Firenze)
- 16.30 Pricing Problems
- 16.31 Divide a Quantity Problems
- 16.32 Guessing a chosen number (MS Verona)
- 16.33 Divisibility of the Numbers 1-112 (MS Ithaca)
- 17 Apparatus
- 18 Appendix: Bibliography
| Arithmetic Book | [1]ספר חשבון | |||||||||||||||||||||||||||||||
The Nature of One |
||||||||||||||||||||||||||||||||
| One, from one aspect, is a number and the foundation of the numbers; from another aspect it is not a number. | האחד מדרך אחד הוא מן המספר ועיקר המספר ומדרך אחר אינו מספר[2] | |||||||||||||||||||||||||||||||
| The proof that indicates that it is not a number is that every number is half [the sum of the numbers on] its both sides and also [the sum of the numbers on] the sides of its both sides and so on until the end of its sides.
|
והאות המורה כי אננו מספר הוא כי כל מספר הוא מחצית שני צדיו וכן הוא מחצית שני צדי צדיו על תכלית כל צדיו[3] | |||||||||||||||||||||||||||||||
|
המשל בזה י' צדו האחד י"א וצדו האחר ט' ושניהם כ' וי' הוא חצי שני הצדדים[4] | |||||||||||||||||||||||||||||||
|
וכן הוא חצי שני צדי צדיו שהם י"ב וח' | |||||||||||||||||||||||||||||||
|
וכן הוא מחצית שני צדי צדי צדיו שהם י"ג וז'[5] | |||||||||||||||||||||||||||||||
| Ans so on, every number is half [the sum of the numbers on] its both sides. | וכן כל המספר הוא מחצית ב' צידיו | |||||||||||||||||||||||||||||||
|
והאחד מפני שאינו מספר אי אתה מוצא לו ב' צדדים[6] | |||||||||||||||||||||||||||||||
|
ומצד היותו מספר הוא מחצית חצי צדו האחד ר"ל חצי מספר ב' | |||||||||||||||||||||||||||||||
| Another proof that indicates that one is not a number is that every number is either integer or a fraction. | ואות אחר מורה שהאחד אינו מספר כי כל מספ' הוא או מספר שלם או שבר מספר[7] | |||||||||||||||||||||||||||||||
|
ומדרך המספר השלם כשתרבה אותו בעצמו יוסיף | |||||||||||||||||||||||||||||||
|
כגון ב' פעמי' ב' ד'[8] | |||||||||||||||||||||||||||||||
|
ג' פעמי' ג' ט'[9] | |||||||||||||||||||||||||||||||
|
וכן כלם | |||||||||||||||||||||||||||||||
|
ושבר מספר כשתרבה[10] אותו בעצמו יחסר | |||||||||||||||||||||||||||||||
|
כגון[11] חצי פעם חצי[12] שהוא רביע | |||||||||||||||||||||||||||||||
|
שליש פעם א' שליש[13] שהוא[14] תשיע | |||||||||||||||||||||||||||||||
|
רביע פעם רביע[15] שהוא[16] חלק מי"ו | |||||||||||||||||||||||||||||||
|
וכן כלם | |||||||||||||||||||||||||||||||
|
והאחד שאינו מוסיף על חשבונו כשתמנהו בעצמו כדרך השלמים ולא גורע [ממניינו][17] כדרך השברים יורה שאיננו[18] מספר | |||||||||||||||||||||||||||||||
Shortcuts - Products of Ranks by Ranks |
||||||||||||||||||||||||||||||||
| Units by Tens | ||||||||||||||||||||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| When we want to multiply units by tens.
|
כשנרצה[19] לרבות[20] אחדים עם עשרות | |||||||||||||||||||||||||||||||
|
כגון ו' פעמי' מ' | |||||||||||||||||||||||||||||||
|
נקח[21] דמיונם[22] מן האחדים הדומים להם ובעבור שמ' הם ד' עשרות מניינם הוא ו' פעמים וכמו[23] שו' פעמי' ד'[24] הם[25] כ"ד כן ו' פעמים מ'[26] הם[27] כ"ד עשרות שהם ר"מ | |||||||||||||||||||||||||||||||
|
וכן[28] ג' פעמים ל'[29] | |||||||||||||||||||||||||||||||
|
דמיונם הוא[30] ג' פעמים ג' שהם ט' ויהיו[31] ט' עשרות שהם צ' | |||||||||||||||||||||||||||||||
| This way for all of them. | ועל זה הדרך הם כלם[32] | |||||||||||||||||||||||||||||||
| Tens by Tens | ||||||||||||||||||||||||||||||||
| If we multiply tens by tens.
|
ואם נרבה[33] עשרות עם עשרות | |||||||||||||||||||||||||||||||
|
כגון ל' פעמים ל' | |||||||||||||||||||||||||||||||
|
ניקח דמיונם מן האחדים הדומים להם ובעבור היות ל'[34] ג'[35] עשרו' דמיונם הוא ג' פעמי' ג'[36] וכמו שג' פעמים ג'[37] הם ט' כן ל' פעמים ל' הם ט' מאות | |||||||||||||||||||||||||||||||
|
וכן מ' פעמים מ'[38] | |||||||||||||||||||||||||||||||
|
דמיונם הוא ד' פעמים שהם י"ו[39] מאות[40] | |||||||||||||||||||||||||||||||
|
וכן ל' פעמים מ'[41] | |||||||||||||||||||||||||||||||
|
דמיונם הוא ג' פעמים ד'[42] שהם י"ב וכן ל' פע' מ'[43] הם י"ב מאות | |||||||||||||||||||||||||||||||
| The same for all. | וכן כלם | |||||||||||||||||||||||||||||||
| The rule for this is that when we multiply units by tens, we take their analogous from the multiplication of the units by the units and the result is tens. | והכלל המסור לזה הוא כשנרבה אחדים עם עשרות נקח דמיונם מרבוי האחדים עם האחדים ויהיו העולה עשרות | |||||||||||||||||||||||||||||||
| If we multiply tens by tens the result is hundreds. | ואם נרבה עשרות עם עשרות יהיה העולה מאות[44] | |||||||||||||||||||||||||||||||
| Tens by Hundreds | ||||||||||||||||||||||||||||||||
| If we multiply tens by hundreds, the result is thousands.
|
ואם נרבה עשרות[45]עם מאות יהיה[46] העולה אלפים | |||||||||||||||||||||||||||||||
|
כגון כ' פעמים ב' מאות[47] | |||||||||||||||||||||||||||||||
|
דמיונם שהם ב' פעמים ב'[48] שהם ד' והם[49] ד' אלפים | |||||||||||||||||||||||||||||||
|
וכן נ' פעמי' ת"ק | |||||||||||||||||||||||||||||||
|
דמיונם ה' פעמי' ה'[50] שהם[51] כ"ה והם[52] כ"ה אלפים | |||||||||||||||||||||||||||||||
| The same for all. | וכן כלם | |||||||||||||||||||||||||||||||
| Hundreds by Hundreds | ||||||||||||||||||||||||||||||||
| If we multiply hundreds by hundreds the result is tens of thousands.
|
ואם נרבה מאות עם מאות יהיה העולה עשרות מאלפים | |||||||||||||||||||||||||||||||
|
כגון ר' פעמים ר'[53] | |||||||||||||||||||||||||||||||
|
דמיונם הם[54] ב' פעמים ב'[55] שהם[56] ד' [57]והם ד' עשרות מאלפים[58] | |||||||||||||||||||||||||||||||
|
וכן ת"ק פעמים ת"ק | |||||||||||||||||||||||||||||||
|
דמיונם ה' פעמים ה'[59] שהם כ"ה והם[60] כ"ה עשרות מאלפים | |||||||||||||||||||||||||||||||
| The same for all. | וכן כלם | |||||||||||||||||||||||||||||||
The Decimal Ranks[61] |
||||||||||||||||||||||||||||||||
| The ranks of numbers one above the other: | מדרגות המספרים זו למעלה מזו | |||||||||||||||||||||||||||||||
|
האחדים הם במדרגה הראשונה | |||||||||||||||||||||||||||||||
|
העשרות הם במדרגה השנית | |||||||||||||||||||||||||||||||
|
המאות הם במדרגה השלישית | |||||||||||||||||||||||||||||||
|
האלפים הם במדרגה הרביעית | |||||||||||||||||||||||||||||||
|
העשרות מהאלפים הם במדרגה החמישית | |||||||||||||||||||||||||||||||
|
המאות מהאלפים הם במדרגה השישית | |||||||||||||||||||||||||||||||
| So they rise from rank to rank. | וכן עולים ממדרגה למדרגה | |||||||||||||||||||||||||||||||
|
ואם נרצה לרבות[62] ת"ר[63] בד' אלפים | |||||||||||||||||||||||||||||||
|
דמיונם ו'פ'ד' שהם[64] כ"ד | |||||||||||||||||||||||||||||||
|
ואם תרצה לדעת אלו הכ"ד מה יורו קח מעלת המאות שהוא שלשה ומנה מן האלפים ג' מעלות והמעלה שתגיע אליה היא מעלת כ"ד ששמרת[65] והיא מעלת מאות האלפים[66] | |||||||||||||||||||||||||||||||
|
וכן אם אתה מונה מעלת האלפים והוא ד' ממעלת המאות אתה מגיע גם כן[67] אל מעלת מאות האלפים | |||||||||||||||||||||||||||||||
|
ותדע מכאן כי האחדים מהמספר אשר שמרת והוא כ"ד הוא ממעלת מאות האלפים ועשרותיו הם ממעלת אלפי האלפים[68] יהיו אם כן[69] כ"ד אשר שמרת ב' אלפי אלפים ות' אלף כי אחדיו הם ארבעה ועשרותיו הם ב' | |||||||||||||||||||||||||||||||
| Another method: consider [the positional values] of the two ranks and know their [sum]. Subtract 1 from it; the remainder is the rank of the product. | דרך אחרת תחשוב אישי [פי' אחדי'] ב' המעלות ותדע מספרם ותפחות מהם א'[70] והנשאר יהיה מעלת הנכלל | |||||||||||||||||||||||||||||||
|
כאלו תקח במשל הזה אוש המאות והוא ג' ואוש האלפים והוא ד' יהיו ז' ותפחות מהם א' וישארו ו' וזה המספר הוא אוש מאות האלפים | |||||||||||||||||||||||||||||||
Three Types of Numbers[71] |
||||||||||||||||||||||||||||||||
| The numbers are [of] three [types]: perfect number, abundant number, deficient number. | המספרים הם ג' מספר שלם מספר עודף מספר חסר | |||||||||||||||||||||||||||||||
| The perfect number, as the number 6, its parts divide it and complete it [= it is equal to the sum of its divisors]; they do not exceed it, nor less than it. Because its parts are a half, a sixth, and a third. If you sum them up, they are six, which is the same as it.
|
מספר שלם הוא כמו מספר ו' אשר חלקיו המונים אותו ממלאים אותו ואינם עודפים עליו ואינם[72] חסרים ממנו כי חלקיו הם חצי ושתות ושליש ואם תקבצם יהיו ששה כמוהו | |||||||||||||||||||||||||||||||
| The same for the number 28 - its parts are: one part of 28, one part of 14, a seventh, a quarter, and a half. If you sum them up, they are 28, which is the same as it. | וכן מספר כ"ח חלקיו הם חלק מכ"ח וחלק מי"ד ושביע ורביע וחצי ואם תקבצם יהיו כ"ח כמוהו | |||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||
| The abundant number, as 12, whose parts are one part of 12, a sixth, a third, a quarter, and a half. If you sum them up, they are 16. | ומספר עודף הוא י"ב שחלקיו הם חלק מי"ב ושתות ושליש ורביע וחצי ואם תקבצם יהיו כלם י"ו | |||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||
| The deficient number, as 14, whose parts are one part of 14, its half, and its seventh. If you sum them up, they are ten.
|
ומספר חסר כגון מספר י"ד שחלקיו הם חלק מי"ד וחציו ושביעו ואם תקבצם יהיו כלם עשר | |||||||||||||||||||||||||||||||
The Ten Numerals[73] |
||||||||||||||||||||||||||||||||
| The sages of India made the shapes of their digits like these: | וחכמי הודו עשו צורות אותיותם כמו אלו | |||||||||||||||||||||||||||||||
| [74]10 9 8 7 6 5 4 3 2 1 | ||||||||||||||||||||||||||||||||
The Method of Finding the Square Numbers[75] |
הסדר למצא המספרים המרובעים | |||||||||||||||||||||||||||||||
| It is that you sum the first square with the odd number that follows it and you will find the second square.
|
הוא שתקבץ המרובע הראשון עם המספר הנפרד הבא אחריו ותמצא המרובע השני | |||||||||||||||||||||||||||||||
|
כמו אם תקבץ האחד שהוא המרובע הראשון עם הג' שהוא ראש[76] הנפרדים[77] תמצא ד' שהוא המרובע השני אשר צלעו ב' | |||||||||||||||||||||||||||||||
|
ואם תקבץ ד' שהוא המרובע השני עם ה' שהוא הנפרד השני יהיו ט' והוא המרובע השלישי אשר צלעו ג' | |||||||||||||||||||||||||||||||
|
ואם אתה מוסיף על ט' שהוא המרובע השלישי המספר הנפרד השלישי שהוא[78] ז' תמצא י"ו שהוא המרובע הרביעי אשר צלעו ד' | |||||||||||||||||||||||||||||||
| This way you find all of them. | ועל זה הסדר תמצאם כלם | |||||||||||||||||||||||||||||||
Cubic Numbers[79] |
||||||||||||||||||||||||||||||||
| Anything whose length, breadth, and depth are equal is called cubic. | כל דבר שארכו ורחבו ועמקו שוים יקרא מעוקב | |||||||||||||||||||||||||||||||
|
והנה א' הוא המעוקב הראשון והוא מקום שארכו א' ורחבו א' וגבהו א' ועמקו א' | |||||||||||||||||||||||||||||||
|
וח' הוא המעקב השני היוצא ממקום שארכו ורחבו ועמקו ב' | |||||||||||||||||||||||||||||||
|
וכ"ז הוא המעוקב השלישי היוצא ממקום שארכו ורחבו ועמקו ג' | |||||||||||||||||||||||||||||||
|
וס"ד הוא המעקב הרביעי היוצא ממקום שארכו ורחבו ועמקו ד' | |||||||||||||||||||||||||||||||
|
וקכ"ה הוא המעוקב החמשי היוצא ממקום שארכו ורחבו ועמקו ועמקו ה' | |||||||||||||||||||||||||||||||
|
ורי"ו הוא המעוקב הששי היוצא ממקום שארכו ורחבו ועמקו ו' | |||||||||||||||||||||||||||||||
|
ושמ"ג הוא המעקב השבעי היוצא ממקום שארכו ורחבו ועמקו ז' | |||||||||||||||||||||||||||||||
|
ותקי"ב הוא המעוקב השמני היוצא ממקום שארכו ורחבו ועמקו ח' | |||||||||||||||||||||||||||||||
|
ותשכ"ט הוא המעוקב התשעי היוצא ממקום שארכו ורחבו ועמקו ט' | |||||||||||||||||||||||||||||||
|
ואלף הוא המעוקב העשרי היוצא ממקום שארכו ורחבו ועמקו י' | |||||||||||||||||||||||||||||||
| You see that every cubic number, [when it is] doubled, is 8 times the original [cubic] number.
|
והנך רואה כי כל מעוקב [זוג] יהיה ח' פעמי' מהמספר הראשון | |||||||||||||||||||||||||||||||
|
כי כל מעקב א' הוא א' | |||||||||||||||||||||||||||||||
|
ומעקב ב' שהוא כפל א' הוא ח' | |||||||||||||||||||||||||||||||
|
ומעקב ד' שהוא כפל ב' הוא ס"ד שהוא ח' פ' במעקב ב' | |||||||||||||||||||||||||||||||
|
וכן מעקב ו' הוא ח' פ' במעקב ג' | |||||||||||||||||||||||||||||||
| And so on. | וכן לעולם | |||||||||||||||||||||||||||||||
| Word Problem - Payment Problem | ||||||||||||||||||||||||||||||||
|
ולכן מי שיאמר חפור לי בור שיהיה ב' ארכו ב' רחבו ב' עמקו יהיה שכרו מ' ליט' שהוא ח' פעמי' ה' ליט' | |||||||||||||||||||||||||||||||
| Because every cubic number, when it is doubled, is 8 times the original [cubic] number. | כי כל מעקב כשיכפול יהיה ח' פעמים מהמספר הראשון | |||||||||||||||||||||||||||||||
Primary Definitions[80] |
||||||||||||||||||||||||||||||||
|
האחדות הוא הענין אשר בו קוראים לכל אחד מהנמצאי' בעולם אחד | |||||||||||||||||||||||||||||||
|
המספר הוא הרבוי הנקבץ מן האחדים | |||||||||||||||||||||||||||||||
|
המספר המנוי במספר אחר הוא מספר הנקבץ מכפל המספר המנוי פעמי' אשר מניינם כמניין האחדים אשר במספר השני אשר הוא נמנה בו | |||||||||||||||||||||||||||||||
|
המספר המרובע הוא המספר הנקבץ מכפל מספר במספר אחדיו הנמצאי' בו באותו המספר עצמו | |||||||||||||||||||||||||||||||
|
המספר הראשון הוא גדר המרובע | |||||||||||||||||||||||||||||||
|
כגון מספר תשעה נקרא מרובע מפני שהוא נקבץ מכפל השלשה שלשה פעמי' | |||||||||||||||||||||||||||||||
|
ומספר השלשה נקרא גדר המרובע | |||||||||||||||||||||||||||||||
|
והמספר מעקב הוא הגוף השוה שארכו ורחבו ועמקו שוים והמספר הזה הוא המספר הנקבץ מכפל מספר המרובע כמנין גדרו | |||||||||||||||||||||||||||||||
|
כגון מספר ט' שהוא 9 מרובע אם אתה כופל אותו במניין ג' שהוא גדרו יהיה הנקבץ מזה כ"ז והמספר הזה הוא הנקרא מספר מעוקב | |||||||||||||||||||||||||||||||
|
הקשת מניין אל מניין או תמונה לתמונה או ערך אחר כן השני | |||||||||||||||||||||||||||||||
Multiplication |
||||||||||||||||||||||||||||||||
Units and tens by units and tens |
||||||||||||||||||||||||||||||||
| The method of multiplying two digits by two digits | דרך רבוי ב' אותיות כנגד ב' אותיות[81] | |||||||||||||||||||||||||||||||
| When we wish to multiply two digits by two digits: | כשנרצה[82] לרבות ב' אותיות כנגד ב' אותיות | |||||||||||||||||||||||||||||||
| We multiply them three times one after another: first, we multiply the units by the units; then we multiply the units by the tens; and then we multiply the tens by the tens. | נרבה אותם ג' פעמי' זה אחר זה בראשונה נרבה האחדים עם האחדים | |||||||||||||||||||||||||||||||
| Here is the example of the [ordered] multiplications: | והנה דמיון הריבוי הקודם והמתאחר | |||||||||||||||||||||||||||||||
|
רצינו לרבות כ"ב פעמים כ"ב | |||||||||||||||||||||||||||||||
| ||||||||||||||||||||||||||||||||
|
נרבה בתחילת האחדים זה עם זה ונאמר ב' פעמים ב' הם ד' ונכתוב ד' למעלה מן האחדים מצד ימין | |||||||||||||||||||||||||||||||
|
אחרי כן נרבה האחדים עם העשרות ונאמר ב' פעמי' ד' וב' פעמים ד' הרי ח' ונכתוב ח' | |||||||||||||||||||||||||||||||
|
אחרי כן נרבה העשרות זו עם זו ונאמר ב' פעמים ב' ד' ונכתוב ד' | |||||||||||||||||||||||||||||||
|
יצא לנו כי כ"ב פעמים כ"ב הם תפ"ד | |||||||||||||||||||||||||||||||
| ||||||||||||||||||||||||||||||||
|
וכן אם רצינו לרבות מ"ד פעמים מ"ד | |||||||||||||||||||||||||||||||
| ||||||||||||||||||||||||||||||||
|
נרבה האחדים זה עם זה בתחילה ונאמר ד' פעמי' ד' הם י"ו ונכתוב ו' ונחזיק[85] בידינו העשרון שהוא א' | |||||||||||||||||||||||||||||||
|
אחר כן נרבה האחדים עם העשרות ונאמר ד' פעמי' ד' י"ו וד' פעמים ד' י"ו הרי ל"ב וצרף עמהם הא' שהחזקת בידך הרי ל"ג ונכתוב ג' ונחזיק בידינו ג' | |||||||||||||||||||||||||||||||
|
אחרי כן נרבה העשרות עם העשרות נאמר ד' פעמים ד' י"ו ועם הג' שהחזקנו בידינו הרי י"ט ונכתוב י"ט | |||||||||||||||||||||||||||||||
|
היוצא מזה כי מ"ד פעמים מ"ד הם אלף ות"תקל"ו | |||||||||||||||||||||||||||||||
| ||||||||||||||||||||||||||||||||
|
וכן אם רצינו לרבות כף פעמים כף | |||||||||||||||||||||||||||||||
| ||||||||||||||||||||||||||||||||
|
נאמר ציפרא פעם ציפרא ציפרא ונכתבנה | |||||||||||||||||||||||||||||||
|
אחר כך נאמר ציפרא פעמים פעם ב' וב' פעמים ציפרא ציפרא ונכתבנה | |||||||||||||||||||||||||||||||
|
ואחר כך נאמ' ב' פעמים ב' הם ד' ונכתבם | |||||||||||||||||||||||||||||||
|
יצא לנו כי כ פעמים כ הם ארבע מאות | |||||||||||||||||||||||||||||||
| ||||||||||||||||||||||||||||||||
Units by units and tens |
||||||||||||||||||||||||||||||||
| If we want to multiply one digit by two digits. | ואם רצינו לרבות אות אחד שנגד ב אותיות | |||||||||||||||||||||||||||||||
|
כגון שרצינו לדעת ז' פעמים מ"ה כמו שתראה בצורה | |||||||||||||||||||||||||||||||
| ||||||||||||||||||||||||||||||||
|
נרבה אותם עם הציפרא כדי שיהיו ב' אותיות כנגד ב' אותיות ויעשה אותם בנקל | |||||||||||||||||||||||||||||||
|
ונאמר ה' פעמי' ז' ל"ה ונכתוב האחדים שהם ה' ונחזיק בידינו העשרות שהם ג' | |||||||||||||||||||||||||||||||
|
אחר כך נאמר ציפרא פע' ה' ציפרא וד' פעמים ז' כ"ח וצרף עמהם הג' שהחזקנו בידינו יהיו ל"א ונכתוב ל"א כי מעתה לא נשאר לנו לרבות דבר אחר | |||||||||||||||||||||||||||||||
|
כי ציפרא פעם ד' שנשאר לנו לרבות הוא ציפרא ואינם עולים לכלום | |||||||||||||||||||||||||||||||
|
יצא לנו כי ז' פעמים מ"ה הם שט"ו | |||||||||||||||||||||||||||||||
| ||||||||||||||||||||||||||||||||
Check |
||||||||||||||||||||||||||||||||
| If we want to check if the number we received is correct: | ואם נרצה לבחון אם החשבון שיצא לנו הוא אמתי | |||||||||||||||||||||||||||||||
|
נעשה על זה הדרך בזה החשבון שהוא כ"ב פעמים כ"ב | |||||||||||||||||||||||||||||||
| ||||||||||||||||||||||||||||||||
|
נקבץ הב' אותיות העליונות ונאמר ב' וב' הם ד' ונכתוב אותם אצל שתי האותיות התחתונות | |||||||||||||||||||||||||||||||
|
אחרי כן נרבה אלו שתי אותיות שכתבנו זו עם זו ונאמר ד' פעמי' י"ו | |||||||||||||||||||||||||||||||
|
נשליך מהם הט' ישארו ז' ונכתוב ז' בתוך שתי האותיות הנזכרות | |||||||||||||||||||||||||||||||
|
וכמו שנשארו בידינו ז' כן צריך שישארו בידינו ז' מן ת'פ'ד' שעולה החשבון אחר שנשליך מהם הט' | |||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||
|
והנה אם נקבץ ד' וח' וד' שהם האותיות המורות ת'פ'ד' יהיו י"ו | |||||||||||||||||||||||||||||||
|
ואם נשליך מהם הט' ישארו | |||||||||||||||||||||||||||||||
|
וכן בחשבון השני שעשינו שהם מ"ד פעמים מ"ד | |||||||||||||||||||||||||||||||
| ||||||||||||||||||||||||||||||||
|
נקבץ [86]האותיות העליונות ונאמר ד' וד' הם ח' ונכתבם | |||||||||||||||||||||||||||||||
|
ונרבה אותם זו עם זו ונאמר ח' פע' ח' הם ס"ד | |||||||||||||||||||||||||||||||
|
השליכם מהם ט' ט' ישאר מהם א' | |||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||
|
וכן צריך להשאר מאלף ת"תק'ל'ו' שסימנם הוא א' וט' וג' וו' | |||||||||||||||||||||||||||||||
|
ואם | |||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||
Units, tens, and hundreds by units, tens, and hundreds |
||||||||||||||||||||||||||||||||
| If we want to multiply three digits by three digits. | ואם נרצה לרבות ג' אותיות כנגד ג' אותיות | |||||||||||||||||||||||||||||||
|
כגון שרצינו לדעת רכ"ב פעמים רכ"ב פעמים | |||||||||||||||||||||||||||||||
|
נרבה אותם ה' פעמים וזהו דרך ריבויים | |||||||||||||||||||||||||||||||
|
ונכתוב אותם ה' פעמים כדי שיתבאר למראית העין הרבוי הראשון והשני והשלישי והרביעי וחמשי | |||||||||||||||||||||||||||||||
| ||||||||||||||||||||||||||||||||
|
ואם נרצה לרבות רכ"ב פעמים רכ"ב | |||||||||||||||||||||||||||||||
| ||||||||||||||||||||||||||||||||
|
נתחיל לרבות האחדים ונאמר ב' פעמים ב' הם ד' ונכתבם | |||||||||||||||||||||||||||||||
|
אחר זה נרבה האחדי' עם העשרות ונאמר ב' פעמי' ב' הם ד' וב' פעמים ב' הם ד' הרי ח' ונכתבם[87] | |||||||||||||||||||||||||||||||
| ||||||||||||||||||||||||||||||||
|
אחר זה נרבה שלשתם ביחד ונאמר ב' פעמים ב' ד' וב' פעמים ב' ד' הרי ח'[88] וב'פעמים ב' ד' הרי י"ב ונכתוב ב' ונחזי' בידינו א' | |||||||||||||||||||||||||||||||
| ||||||||||||||||||||||||||||||||
|
אחר זה נרבה העשרות עם המאות ונאמר ב' פעמים ב' ד' וב' פעמי' ב' ד' הרי ח' וא' שהחזקנו בידינו הרי ט' ונכתבם | |||||||||||||||||||||||||||||||
|
אחר זה נרבה המאות עם המאות ונאמר ב' פעמי' ב' ד' ונכתבם | |||||||||||||||||||||||||||||||
|
יצא לנו כי רכ"ב פעמים רכ"ב הם מ"ט אלפים ורפ"ד | |||||||||||||||||||||||||||||||
| ||||||||||||||||||||||||||||||||
Check |
||||||||||||||||||||||||||||||||
| To check if the calculation is correct: | ולבחון אם החשבון אמת | |||||||||||||||||||||||||||||||
| ||||||||||||||||||||||||||||||||
|
קבצנו רכ"ב העליונים שסימנם ב'ב'ב' ועלו ו' וכתבנום אצלם | |||||||||||||||||||||||||||||||
|
רבינו ו' על ו' ועלו ל"ו | |||||||||||||||||||||||||||||||
|
השלכנו אותם ט'ט' ולא נשאר בידינו ונתננו ציפרא באמצעם | |||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||
|
וכן צריך להשאר הציפרא מן הסכום מן היוצא מן החשבון שהם מ"ט אלפים ורפ"ד אשר דמיונם הם ד' ט' ב' ח' ד' ועולה כ"ז[89] | |||||||||||||||||||||||||||||||
|
ואם תשליכם ט"ט ישאר ציפרא | |||||||||||||||||||||||||||||||
|
נמצא שהחשבון הוא אמת | |||||||||||||||||||||||||||||||
Units and tens by units, tens, and hundreds |
||||||||||||||||||||||||||||||||
| If we want to multiply two digits by three [digits]. | ואם רצינו לרבות ב' אותיות כנגד ג' | |||||||||||||||||||||||||||||||
|
כגון שרצינו לומר | |||||||||||||||||||||||||||||||
| ||||||||||||||||||||||||||||||||
|
נשוה אותה עם הציפרא כדי שיהיו ג' אותיות כנגד ג' אותיות ויקל עשייתו | |||||||||||||||||||||||||||||||
Units by units, tens, and hundreds |
||||||||||||||||||||||||||||||||
| If we want to multiply one digit by three [digits]. | וכן אם רצינו לרבות אות[91] אחת כנגד שלשה | |||||||||||||||||||||||||||||||
| We match them also by [adding] zeros. | נשוה אותה עם הציפרא ג"כ | |||||||||||||||||||||||||||||||
|
כגון שרצינו לדעת ט' פעמים ר'כ'ב' | |||||||||||||||||||||||||||||||
|
נכתוב אותם בזה הדרך כאשר תראה בצורה | |||||||||||||||||||||||||||||||
| ||||||||||||||||||||||||||||||||
Units, tens, hundreds, and thousands by units, tens, hundreds, and thousands |
||||||||||||||||||||||||||||||||
| If we want to multiply four digits by four digits. | אם[92] נרצה לרבות ד'[93] אותיות כנגד ד'[94] אותיות | |||||||||||||||||||||||||||||||
|
כג כגון שרצינו לרבות ב' אלפים ור'כ'ב' פעמי' ב' אלפים ור'כ'ב' | |||||||||||||||||||||||||||||||
|
נרבה אותם ז' פעמים ונכתוב אותם ז' פעמי'[95] כדי שיתבאר למעיין הקודם והמתאחר[96] | |||||||||||||||||||||||||||||||
| ||||||||||||||||||||||||||||||||
|
ואם רצינו לרבות ב' אלפי' ור'כ'ב' עם ב' אלפי' ור'כ'ב' | |||||||||||||||||||||||||||||||
| ||||||||||||||||||||||||||||||||
|
נעשה כאשר עשינו בחשבונו' הקודמי' | |||||||||||||||||||||||||||||||
|
ויעלה החשבון ד' אלפי אלפים ות'ת'ק'ל'ז' אלפי' ור'פ'ד' | |||||||||||||||||||||||||||||||
Units, tens, hundreds, and thousands by units, tens, and hundreds |
||||||||||||||||||||||||||||||||
| If we want to multiply four digits by three digits. | ואם רצינו לרבות ד' אותיות כנגד ג' אותיות | |||||||||||||||||||||||||||||||
|
כגון שרצינו לרבות ב' אלפים ור'כ'ב' כנגד ר'כ'ב' | |||||||||||||||||||||||||||||||
|
נשוה אותם עם הציפרא כאשר תראה בצורה | |||||||||||||||||||||||||||||||
| ||||||||||||||||||||||||||||||||
|
ועולים ת'צ'ג' אלפים ור'פ'ד' | |||||||||||||||||||||||||||||||
Units, tens, hundreds, and thousands by units and tens |
||||||||||||||||||||||||||||||||
| If we want to multiply two digits by four [digits]. | וכן אם רצינו לרבות ב' אותיות כנגד ד' | |||||||||||||||||||||||||||||||
|
כגון שרצינו לרבות ב' אלפים ור'כ'ב' כנגד כ"ב | |||||||||||||||||||||||||||||||
|
נשוה אותם עם הציפרא כאשר תראה בצורה | |||||||||||||||||||||||||||||||
| ||||||||||||||||||||||||||||||||
|
ועולים מ"ח אלפים ות'ת'פ'ד' | |||||||||||||||||||||||||||||||
Units, tens, hundreds, and thousands by units |
||||||||||||||||||||||||||||||||
| If we want to multiply one digit by four digits. | וכן אם רצינו לרבות אות אחד כנגד כנגד ד' אותיות | |||||||||||||||||||||||||||||||
|
כגון ב' עם ב' אלפים ור'כ'ב' | |||||||||||||||||||||||||||||||
|
נשוה אותם עם הציפרא כאשר תראה בצורה | |||||||||||||||||||||||||||||||
| ||||||||||||||||||||||||||||||||
|
ועולים ד' אלפים ות'מ'ד' | |||||||||||||||||||||||||||||||
Units, tens, hundreds, thousands, and tens of thousands by units, tens, hundreds, thousands, and tens of thousands |
||||||||||||||||||||||||||||||||
| If we want to multiply five digits by five digits. | אם נרצה לרבות ה' אותיות כנגד ה' אותיות | |||||||||||||||||||||||||||||||
|
כגון שרצינו לדעת כ"ב אלפים ור'כ'ב' כ"ב אלפי' ור'כ'ב' | |||||||||||||||||||||||||||||||
|
נרבה אותם ט' פעמים וזה דרך רבויים | |||||||||||||||||||||||||||||||
|
ונכתוב אותם ט' פעמי' כדי שיתבאר למעיין הקודם הקודם והמתאחר | |||||||||||||||||||||||||||||||
| ||||||||||||||||||||||||||||||||
Units, tens, hundreds, thousands, tens of thousands, and hundreds of thousands by units, tens, hundreds, thousands, tens of thousands, and hundreds of thousands |
||||||||||||||||||||||||||||||||
| If we want to multiply six digits by six digits. | ואם נרצה לרבות ו' אותיות כנגד ו' אותיות | |||||||||||||||||||||||||||||||
| We multiply them 11 times. | נרבה אותם י"א פעמי' | |||||||||||||||||||||||||||||||
|
כגון שרצינו לרבות ר'כ'ב' אלפי' ור'כ'ב' ר'כ'ב' אלפים ור'כ'ב' | |||||||||||||||||||||||||||||||
|
ונרבה אותם י"א פעמי' כדי שיתבאר למעיין הקודם והמתאחר | |||||||||||||||||||||||||||||||
|
The Method of Summing Up Separate Numbers Called Congregare or Riunire |
דרך קיבוץ חשבונות נפרדים הנקראים קונגרייג או ריונרייי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If we want to sum up separate numbers and bring them into one sum, we do this way: we write them successively one beneath the other: the units beneath the units, the tens corresponding the tens, the hundreds corresponding the hundreds and the thousands corresponding the thousands. | אם רצינו לקבץ חשבונות נפרדים ולהביאם לסכום אחד נעשה על זאת הדרך שנכתוב אותם על הסדר זה תחת זה האחדי' תחת האחדים והעשרות נכח העשרות והמאות נכח המאות והאלפים נכח האלפים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| We start summing the units and write [their sum]. | ונתחיל לקבץ האחדי' ולכותבם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If [the sum] is more than ten, we write the units and keep the tens. | אם יעלו על עשר נכתוב האחדים ונחזיק בידינו העשרות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Then, we sum the hundreds, then the thousands, as you see in this diagram: | אחרי כן נקבץ המאות אחרי כן נקבץ האלפים כמו שתראה בצורה הזאת | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
[97]דמיון זה רצינו לקבץ י"ב[98] אלפים וש'מ'ה' וס"ז אלפי' ות'ת'צ'א' וכ"ג אלפים ת'נ'ו' וע"ח אלפים ות'ת'ק'י'ב' ול"ד אלפים ות'ק'ס'ז'[99] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נתחיל לקבץ האחדים ונאמר ה' וא' וו' וב' וז' הם כ"א נכתוב א' ונחזי' בידינו ב' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נקבץ אחרי כן העשרות ונאמר ד' וט' וה' וא' וו' הם כ"ה וב' שהחזקנו בידינו הם כ"ז נכתו' ז' ונחזיק בידינו ב' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נקבץ אחרי כן המאות ונאמ' ג' וח' וד' וט' וה' הם כ"ט וב' שהחזקנו בידינו הרי ל"א נכתוב א' ונחזיק בידינו ג' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נקבץ אחרי כן האלפים ונאמר ב' וז' וג' וח' וד' הם כ"ד וג' שהחזקנו בידינו הרי כ"ז ונכתוב ז' ונחזיק בידינו ב' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נקבץ אחר זה העשרות מן האלפים ונאמ' א' וו' וב'[100] וז' וג' הם י"ט[101] וב' שהחזקנו בידינו הרי כ"א נכתוב אותם כלם כי אין לנו לקבץ חשבון אחר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עלו כלם רי"ז אלפים וקע"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If we want to sum up numbers, some of which have three digits, some have four, some have two, and some have more or less: | ואם רצינו לקבץ חשבונות נפרדים שקצתם הם מג' אותיות וקצתם מד' וקצתם מב' וקצתם מיותר או פחות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| We always write the units corresponding to the units, the tens corresponding to the tens and so on and we match them by [adding] zeros to make the procedure easier. | נכתוב לעולם האחדים נוכח האחדי' והעשרות נוכח העשרות וכן כלם ונשוה אותם עם הציפרי כדי שיקל העניין | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה רצינו לקבץ י"ב אלפים וש'מ'ה' וו' אלפים ות"שפ"ט וקכ"ג ומ"ה אלפים ות"ר ופ' אלפי' ות"תקי"ב וג' אלפים ות"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
התחלנו לקבץ האחדים ואמרנו ה' וט' וג' וב' וו' הם כ"ה כתבנו כ"ה והחזקנו בידינו ב' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
קבצנו אחר כן[102] העשרות ואמרנו ד' וח' וב' וא' הם ט"ו ועם הב' שהחזקנו בידינו הם י"ז כתבנו ז' והחזקנו בידינו א' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
קבצנו אחרי כן המאות ואמרנו ג' וז' וא' וו' וט' וד' הם ל' ועם הא' שהחזקנו בידינו הם ל"א כתבנו א' והחזקנו בידינו ג' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
קבצנו אחרי כן האלפים ואמרנו ב' וו' וה' וג' הם י"ו ועם הג' שהחזקנו בידינו הם י"ט כתבנו ט' והחזקנו בידינו א' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
קבצנו אחר זה העשרות מן האלפי' ואמרנו א' וד' וח' הם י"ג ועם א' שהחזקנו בידינו הם י"ד[103] וכתבנו י"ד כי מעתה קבצנו הכל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועולה סכומם קמ"ט אלפים וקע"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
The Method of Subtracting a Number from a Number Called Sottrarre |
דרך הוצאת מספר ממספר הנק' סורטראיירי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If we want to subtract a number from a number: | אם רצינו לגרוע מספר ממספר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If all the [digits] of the larger number, from which you want to subtract another number, are greater than the [digits] of that number, the procedure is easy. | אם המספר הגדול אשר אתה רוצה לגרוע ממנו מספר אחר כל חלקיו גדולי' מחלקי המספר הוא דרך נקל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה רצינו לגרוע מתת"קפ"ז אלפים ותרנ"ד ת"רמ"ה אלפים ורל"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נעשה על זה הדרך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נתחיל מן האחדים ונאמר מד' הוצא ב' ישארו ב' ונכתוב ב' תחתיהם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומן ה' הוצא ג' ישארו ב' ותכתוב ב' תחתיהם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומן ו' הוצא ב' ישארו ד' ותכתוב ד' תחתיהם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומן ז' תוצא ה' ישארו ב' ונכתוב ב' תחתיהם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומן ח' הוצא ד' ישארו ד' ונכתוב ד' תחתיהם[104] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומן ט' הוצא ו' ישארו ג' ותכתוב ג' תחתיהם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הרי שאם נוציא מת"תק'פ'ז' אלפים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Check - addition: If you want to check if your calculation is correct, sum the digits you subtracted with the digits that remained and if [their sums] are the same as the digits from which you subtracted the other digits, it is correct. | ואם תרצה לבחון אם החשבון שעשית הוא אמתי תקבץ המספרים שהוצאת עם המספרים שנשארו ואם יסכימו עם המספרים שהוצאת המספרים האחרי' ההם הוא אמתי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה קבצנו ב' עם ב' והיו ד' וקבצנו ג' וב' והיו ה' וקבצנו ב' עם ד' והיו ו' וקבצנו ה' עם ב' והיו ז' וקבצנו ד' עם ד' והיו ח' וקבצנו ו' עם ג' והיו ט' והם מסכימים עם המספרים הגדולים אשר הוצאת ממספר הקטנים מהם וזה יורה כי החשבון הוא אמתי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If you want to subtract a number from a number, but some of the digits of the smaller number that you want to subtract from the greater number are greater than some of the [corresponding] digits of the greater number, you should use another procedure: | אמנם אם תרצה לגרוע מספר ממספר ויהיו קצת חלקי המספר הקטן שאתה רוצה לפחות מהמספר הגדול גדולים מקצת מספר חלקי המספר הגדול אתה צריך לעשות על דרך אחרת | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה רצינו לגרוע מצ"ג אלפים ותל"ב כ"ז אלפים ות"רנ"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נתחיל מן האחדי' ותאמר מב' תוצי' ד' לא נוכל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נפחות א' מן הג' שבצדה ונחבר אותה אל הב' ויהיו י"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונאמר מי"ב הוצא ד' ישארו ח'[105] ונכתבם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונחזור אל הג' שפחתנו ממנו א' וישארו ב' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונאמר מב' הוצא ה' לא נוכל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נפחות א' מהד' אשר בצדה ונאמר מי"ב הוצא ה' ישארו ז' ונכתבם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונחזור אל הד' שפחתנו ממנה א' ונשארו ג' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונאמר מג' הוצא ו' ולא נוכל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נפחות א' מן הג' אשר בצדה ונאמר מי"ג הוצא ו' וישארו ז' ונכתבם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונחזור אל הג' שפחתנו ממנו א' ונשארו ב' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונאמר מב' הוצא ז' לא נוכל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נפחות א' מן הט' אשר בצדה ונאמר מי"ב הוצא ז' ישארו ה' ונכתבם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונחזור אל הט' אשר פחתנו ממנה א' ונשארו ח' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונאמר מח' הוצא ב' ישארו ו' ונכתבנו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הרי שהוצאנו מצ"ג אלפים ותל"ב כ"ז אלפים ות"רנ"ד[106] ונשארו ס"ה אלפים ות"שע"ח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Check - addition: If you sum what you subtracted from the mentioned number with the remainder, it will be the same as the original number. | והנה אם תקבץ מה שהוצאתו מהמספר הנזכר עם הנשאר יהיה כמספר הראשון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה שאם נקבץ ד' וח' יהיו י"ב ונכתוב ב' ונחזיק בידינו א' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם נקבץ ה' וז' יהיו י"ב ועם הא' שנשארה בידינו יהיו י"ג ונכתוב ג' ונחזיק א' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם נקבץ ו' וז' יהיו י"ג וא' שנשאר בידינו הרי י"ד ונכתוב ד' ונחזיק א' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם נקבץ ז' וה' יהיו י"ב וא' שנשאר בידינו הרי י"ג ונכתוב ג' ונחזיק א' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם נקבץ ב' וו' יהיו ח' וא' שנשאר בידינו הרי ט' ונכתבם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הרי שהמספר האחרון שוה עם הראשון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The method of division. | דרך חילוק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
The Method of Dividing a Number by a Number Called Dividere or Partire |
[107]דרך חילוק מספר על מספר הנק' דיבי' או פארטיר' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If we want to divide a larger number by a smaller number: | אם נרצה לחלוק מספר גדול על מספר קטן | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If all the digits of the large number are larger than the number by which you want to divide them, the procedure is easy. | אם כל חלקי המספר הגדול הם גדולי מהמספר שאתה רוצה לחלק אותם בו הוא דרך נקל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה רצינו לחלק צ"ח אלפים ות'ש'נ'ו' על ד' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נעשה בזה הדרך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נתחי' מן המספר הגדול ונאמר נחלק ט' על ד' יהיו ב' ונכתבם וישאר בידינו א' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואותו הא' נחבר עם הח' הסמוך לו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונאמר נחלק י"ח על ד' יהיו ד' ונכתבם וישארו בידינו ב' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואלו הב' נחבר עם הז' הבא אחריו ויהיו כ"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונחלקם על ד' ויהיו ו' ונכתבם וישארו בידינו ג' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואילו הג' נחבר עם הבא אחריו ויהיו ל"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונחלקם על ד' יהיו ח' ונכתבם וישארו בידינו ג' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואילו הג' נחבר עם ו' הבא אחריו ויהיו ל"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונחלקם על ד' ויהיו ט' ונכתבם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הרי שחלקנו צ"ח אלפים ותשנ"ו על ד' ועלו כ"ד אלפי' ות"רפ"ט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If we had 1, or 2, or 3 left at the end, they were parts of the 4 by which we divided the number. | ואם היה נשאר בידינו באחרונה א' או ב' או ג' היו חלקי' מד' שחלקנו עליו החשבון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן אלו רצינו לחלק החשבון ח' אלפים ותת"קע"ו על ה' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נעשה על זה הדרך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונאמר חלק ח' על ה' יהיה א' ונכתבנו ישאר ג' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואילו הג' נחבר עם הט' הבא אחריו ויהיו ל"ט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נחלקם על ה' יהיו ז' ונכתבם וישארו ד' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואלו הד' נחברם עם הז' הבא אחריו יהיו מ"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נחלקם על ה' ויהיו ט' ונכתבם וישארו בידינו ב' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואלו הב' נחברם עם ו' הבא אחריו ויהיו כ"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נחלקם על ה' ויהיו ה' ונכתבם וישאר בידינו א' ונכתבנו על ה' אשר חלקנו עליו החשבון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ויהי היוצא מן החלוקה הנזכר' אלף ות"שצ"ה וא' חומש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If we want to divide a number by a number and the digits of the number you want to divide are smaller than the number by which you want to divide them, you should do this way: | אמנם אילו רצינו לחלק מספר על מספר וחלקי המספר שאתה רוצה לחלק קטנים מהמספר שתרצה לחלקם בו אתה צריך לעשות על זה הדרך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה רצינו לחלק י"ב אלפי' ושמ"ה על ט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמרנו חלק א' על ט' לא נוכל[108] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נחבר א' עם הב' הבא אחריו ויהיו י"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקם על ט' יהיה א' ונכתבנו וישארו בידינו ג' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונחברם עם הג' הבא אחריו ויהיו ל"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקם בט' ויהיו ג' ונכתבם וישארו בידינו ו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונחבר אלו הו' עם הד' הבא אחריו ויהיו ס"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקם בט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נחבר הא' עם הה' הבא אחריו יהיו ט"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונאמר חלק ט"ו בט' יהיו א'[109] וישארו בידינו ו' ונכתוב אותם על[110] הט' אשר בו חלקנו כל[111] החשבון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והיוצא מזה הוא שאם נחלק י"ב אלפים וש'מ'ה' על ט' יהיה היוצא מן החלוקה[112] אלפים וש'ע'א' וו' תשיעיות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Check: If you want to check if our calculation is correct, we do as follows: | ואם תרצה לבחון אם החשבון שעשינו הוא אמתי נעשה על זה הדרך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| We sum up the digits of the dividend, cast out the nines and keep the remainder. | היינו שנקבץ המספרים המחולקים ונשליכם ט' ט' ומה שנשאר נחזיק בידינו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Then, we sum up [the digits of] the result of division, cast out the nines and multiply what we received by the divisor. | אחר כן נקבץ מה שעלה בידינו בחלוק ונשליכם ט' ט' ומה שעלה בידינו נרבה אותו עם המחלק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| We cast out the nines from the product we received. | ומה שעלה בידינו מהרבוי נשליכם ט' ט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If the calculation is correct, we receive the same as what we received when we summed the digits of the dividend. | ואם החשבון הוא אמתי יעלה בידינו כמו שעלה בידינו בקבצנו המספרים המחולקים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה הדמיון נעשו בחשבון שכתבנו לעיל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
היינו בתחלה נקבץ המספרי' המחולקים ונאמ' ו' וה' ז' וח' וט' בין כלם ל"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נשליכם ט' ט' ישארו ח' ונכת' ונכתבם אצל המספרי' המחולקים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אחר כן נקבץ מה שעלה בחלוק ונאמ' ט' וח' וו' וד' ב' בין כלם כ"ט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נשליכם ט' ט' וישארו ב' ונכתבם אצל מה שעלה בחלוק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אחר כן נרבה אותו עם המחלק ונאמ' ב' פעמי' ד' ח' והנה עלה בידינו מה שעלה בקבצנו המספרי' המחולקים אם כן החשבון אמתי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If 1, or 2, or 3 remains from the division [procedure], we do this way: | ואם ישארו מן החלוק א' או ב' או ג' נעשה על זה הדרך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| After we sum up [the digits of] the result of division and cast out the nines, we add [what remains] to what we have left [= to the remainder from the division]. | וזה שקבצנו אחר מה שעלה בחלוק והשלכנו אותם ט' ט' נקבצם עם מה שנשאר בידינו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If the calculation is correct, what we receive is the same as what we received when we summed the digits of the dividend. | ואם החשבון אמתי יעלה בידינו כמו שעלה בידינו בקבצנו המספרים המחולקים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה הדמיון נעשו כחשבון השני שכתבנו למעלה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
היינו בתחלה נקבץ המספרי' המחולקים ונאמר ו' וז' וח' וט' בין כלם ל' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נשליכם ט' ט' וישאר בידינו ג' ונכתבנו אצל המספרי' המחולקים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אחר כן נקבץ מה שעלה בידינו בחלוק ונאמר ה' וט' וז' וא' בין כלם כ"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נשליכם ט' ט' וישאר בידינו ד' ונכתבנו אצל מה שעלה בחלוק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אחר כן נרבה אותו עם המחלק ונאמ' ד' פעמי' ה' הם כ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונשליכם ט'ט' וישאר בידינו ב' ונחבר עם הב' הא' שעלה בידינו מן החלוק הכתוב על ה' ויהיו ג' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה שעלה בידינו כמו שעלה בידינו בקבצנו המספרים אם כך הוא החשבון אמתי[113] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Another method of dividing a number by a number | דרך מספר על מספר בדרך אחרת | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| If we want to divide a known number by another number and it is difficult for us to divide it by this number, we divide it twice and we receive as if we divided it once by that number. | [114]אם נרצה לחלק מספר ידוע על מספר אחר ויקשה עלינו לחלקו על המספר ההוא נחלק אותו ב' פעמים ויעלה בידינו כאלו חלקנוהו פעם אחת על המספר ההוא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה רצינו לחלק פ"ט אלפים ות"שס"ה על י"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה אם חלקנוה על י"ב בפעם אחת יעלה ז' אלפים ות"פ וה' חלקים מי"ב וזה סדר עשייתו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמרנו חלק ח' על י"ב לא נוכל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נחבר אל ח' הט' הבא אחריו יהיו פ"ט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקם בי"ב יהיו ז' ונכתבם וישאר בידינו ה' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונחבר אליה ז' הבא אחריו ויהיו נ"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקים בי"ב יהיו ד' ונכתבם וישארו בידינו ט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונחבר אליהם ו' הבא אחריו יהיו צ"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקים בי"ב יהיו ח' ונכתבם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונאמר חלק ה' על יב לא נוכל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותכתוב ציפרא תחת הה' וה' תכתוב על י"ב יהיו ז' אלפים ותש"פ וה' חלקים מי"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם נרצה לחלק זה החשבון בעצמו בעניין אחר נחלק אותו על ג' פעם אחת והעולה נחלק אותו על ד' ויהיה נחלק לי"ב חלקים ג"כ | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונכתוב זה החשבון שנית ונחלקנו על ג' בתחילה ואחר זה נחלקנו על ד' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונאמר ח' חלק אותו על ג' יהיו ב' ונכתבם וישארו ב' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונחבר[115] אותם עם ט' הבא אחריו ויהיו כ"ט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקם בג' יהיו ט' ונכתבם וישארו ב' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונחברם עם הז' הבא אחריו ויהיו כ"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונחלקם על ג' יהיו ט' ונכתבם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונאמר אחר זה ו' חלק בג' יהיו ב' ונכתבם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונאמר אחר זה ה' חלק בג' יהיו א' ונכתבנו וישארו ב' ונכתבם על הג' אשר בו חלקנו זה המספר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אחר זה נחלק זה המספר שעלה בידינו מן החלוקה על ד' ונאמר חלק ב' בד' לא נוכל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חבר ב' עם הט' הבא אחריו יהיו כ"ט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקם בד' יהיו ז' ונכתבם וישאר בידינו א'[116] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונחבר א' עם הט' הבא אחריו יהיו י"ט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקם בד' יהיו ד' ונכתבם וישארו בידינו ג' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונחברם עם הב' הבא אחריו ויהיו ל"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונחלקם בד' יהיו ח' ונכתבם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונחלק א' בד' ולא נוכל ונכתוב ציפרא[117] תחת הא' והא' שלא נוכל לחלקה בד' נכתוב אותה על הד' אשר עליה נחלק החשבון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הרי שחלקת החשבון בג' וד' והוא כאלו חלקתו בי"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותרבה אחר זה הא' שעל הד' עם הג' ואמור א' פעם ג' ג' וב' שעל הג' הרי ה' והם ה' חלקים מי"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
היוצא מדברינו שאם נחלק בראשונ' על ג' והיוצא נחלק על ד' על הדרך שעשינו יעלה מן החלוקה ז' אלפי' ות"פ וה' חלקים מי"ב כאשר עלה בידינו בעת שחלקנוהו פעם אחת על י"ב וזה מבואר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
The Method of Knowing the Numbers Summed One after the Other Successively |
[118]דרך ידיעת המספרים המקובצים זה אחר זה על הסדר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם יאמר אדם א' וב' וג' וד' וה' וו' וז' וח' וטי' וי' כמה שוי בין כלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| You should understand, if the last term is an even number as this number or similar to it, always add one to the last term and multiply it by half the last term.
|
יש לך להבין אם המספר האחרון הוא מספר זוג כמו זה מספר והדומה לו תוסיף מספר אחד לעולם על הי' המספר האחרון ותרבה אותו על חצי המספר האחרו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואמור החצי מי' הוא ה' ותרבה אותו על י"א ה' פעמי' י"א נ"ה וכן יהיו המספרים הנזכרי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם הלך בשאלתו עד י"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור ו'[119] פעמי' י"ג ע"ח[120] יהיו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם הלך בשאלתו עד כ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור י' פעמים כ"א וכן יהיו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם הלך עד מאה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור נ' פעמים ק"א וכן יהיו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Always calculate this way when the last term is even. | ואתה תחשוב לעולם בזה הדרך כשיהיה מספר האחרון זוג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If the last term is an odd number, multiply the greater half of this last term by the whole last term.
|
ואם יהיה מספר האחרון מספר נפרד תרבה הרוב מהמספר ההוא האחרון על כל המספר האחרון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כגון האומר הלכתי בחשבוני עד ז' כמה יהיו כלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור הרוב מז' הם ד' תרבה אותם על | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן אם הלך בשאלתו עד ט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור הרוב מט' הוא ה' מנה אותם בט' יהיו מ"ה וכן יהיו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם הלך עד י"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תרבה ט' על[121] י"ז וכן יהיו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם הלך עד י"ט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תרבה י' עם י"ט וכן | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Sum of Evens |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If he says: I summed all the even numbers successively. | ואם יאמר קבצתי מספרים כלם זוגות על הסדר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כמו ב' וד' וו' וח' וי' וי"ב כמה יהיה בין כלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Multiply half the last term by the number that follows this half. | תרבה החצי מן המספר האחרון על המספר אשר אחרי החצי ההוא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואמור החצי מי"ב הוא ו' והמספר שהוא אחר ו' הוא ז' ו' פעמים ז' הם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם אומר הלכתי עד עשרי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור החצי מעשרי' הוא י' והמספר שאחר י' הוא י"א י' פעמים הם ק"י וכן יהיו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Always calculate this way. | ועל זה הדרך תחשוב לעולם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Sum of Odds |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If he says: I summed all the odd numbers successively. | ואם יאמר קבצתי מספרים כלם נפרדי' על הסדר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כגון א' וג' וה' וז' וט' כמה הם בין כלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Take the greater half of the last term and multiply it by itself. | קח הרוב מהמספר האחרון ותרבה הרוב בעצמו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואמור הרוב מט' הוא ה' וה' פעמים ה' הוא כ"ה וכן יהיו כלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם הלך בשאלתו עד י"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור ו' פעמי' ו' וכן יהיו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם הלך עד י"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור ז' פעמי' ז' וכן יהיו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Always calculate this way. | ועל זה הדרך תחשוב לעולם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Sum of Powers of Two |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If a man says: I summed the doubles to each other.
|
ואם יאמר אדם קבצתי מספרים כפולי' זה על זה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כגון א' וב' וד' וח' וי"ו ול"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| He starts with whichever [number] he wants to start and ends with whichever number he wants to end: double the last term and subtract the first term.
|
בכל מקום שירצה להתחיל יתחיל ובכל חשבון שיחפוץ לסיים יסיים כפול המספר האחרון וחסר המספר הראשון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה האומר קבצתי מספרים כפולי' מא' ועד ל"ב כמה הם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואתה כפול ל"ב שהוא המספר האחרון ויהיו ס"ד וחסר המספר הראשון שהוא א' וישארו ס"ג[122] וכן יהיו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם יאמ' התחלתי מא' וכפלתי עד ס"ד[123] כמה יהיו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפול | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם יאמר התחלתי מאחד וכפלתי עד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפול קכ"ח יהיו רנ"ו חסר א' יהיה רנ"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם יאמר התחלתי מד' וכפלתי עד ל"ב כמה יהיו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפול ל"ב יהיה ס"ד חסר ד' יהיו ס' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The procedure is to double the last and subtract the first. | והחשבון המסור לזה לכפול הא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Sum of Powers of Three |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If a man asks about [the sum of] the triples to each other.
|
[124]ואם ישאל אדם על מספרי' משולשים זה על זה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כגון א' ג' ט' כ"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| He adds to the last its half after subtracting the first, with which he starts, then adds the first to it. | יוסיף על האחרון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דהיינו שיגרע מכ"ז שהוא המספר האחרון המספר הראשון שהוא א' ישארו כ"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
יאמר כ"ו וחציו שהוא י"ג הם ל"ט וא' שהוא החשבון הראשון הרי מ' וכן הם א'ג'ט' כ"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Sum of Powers of Four |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| If he asks about the quadruples.
|
ואם ישאל על מספרי מרובעים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כגון א' ד' י"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| He adds to the last its third after subtracting the first, then adds the first to it.
|
יוסיף על האחרון שליש אחר גרעון הראשון ועוד יוסיף הראשון עמו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה א' ד' י"ו הם כ"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
המספר האחרון הוא י"ו חסר המספר הראשון א שהוא א' ישארו ט"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הוסף על ט"ו שלישו שהוא ה' יהיו כ' הוסף עליהם המספר יהיו כ"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| And so on. | וכן לעולם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The rule:
|
והכלל המסור לזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| For the triples he adds a half.
|
למשולש יוסיף החצי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| For the quadruples he adds a third.
|
ולמרובע יוסיף השלישי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| For the quintuples he adds a quarter.
|
ולמחומש יוסיף | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| For the sextuples he adds a fifth.
|
ולמשושה יוסיף החומש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| It is always done this way. | ועל הדרך הזה יעשה לעולם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Motion Problems - Pursuit |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם יאמר אדם אחד הולך בכל יום ויום י' מילי' ואדם אחר הולך ביום אחד א' מיל וביום ב' ב' מילי' וביום ג' ג' מילים וביום ד' ד' מילי' וכן מוסיף והולך בכל יום ויום | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אתה כפול הי' מילי' שמהלך אותו שמהלך בכל יום ויום י' מילין ויהיו כ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תפחות מהם א' ישארו י"ט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נמצא שבי"ט יעמדו בשוה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שצד אותו שמהלך בכל יום י' מילי' בי"ט ימים יהלך ק"צ מילי' כמניין י' על י"ט שהם ק"צ | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואותו שמוסיף בכל יום מיל אחד בי"ט ימים יהלך ג"כ ק"צ מילין כיצד תפוש הרוב מי"ט והם י' ותכפול אותו על י"ט ויהיה ק"צ | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Always double the [number] of miles that the one who walks the same distance [each day] walks, then subtract one from it and this is the number of days, in which they walk the same [total distance].
|
וכן לעולם תכפול המילי' שמהלך המילי' שמהלך הקבוע ותפחות מהם אחד וכמספרם יהיו הימים שיעמדו בשוה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם יאמר אדם אחד הולך בכל יום י' מילי' ואדם[125] אחר הולך ביום אחד ב' מילין וביום שני ד' וביום שלישי ו' וביום רביעי ח' וכן לעולם הוא מוסיף ב' והולך על הסדר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אתה תפחות אחת מן המילין שמהלך הקבוע בכל יום ישאירו ט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נמצא שבט' ימים יעמדו בשוה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כיצד אותו שמהלך בכל יום י' מילין בט' ימים מהלך צ' מילי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואותו שמהלך זוגות ר"ל ב' וד' וו' וח' וי' וי"ב וי"ד וי"ו וי"ח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור החצי מי"ח הם ט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תרבה אותם עם י' שהוא החשבון הבא אחר ט' יהיו צ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נמצא שבט' ימים יעמדו בשוה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Always subtract [one] from the [number] of miles that the one who walks the same distance [each day] walks and this is the number of days, in which they walk the same [total distance].
|
וכן לעולם תפחות מן המיל' שמהלך הקבוע וכמספרם יהיו הימים יעמדו בשוה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם יאמר אדם אחד הולך בכל יום י' מילי' ואדם אחר הולך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Know that the number of miles that the one who walks the same [distance] [each day] walks each day is the same as the number of days, in which they walk the same [total distance].
|
דע כי כמספר המילין מהלך ק הקבוע בכל יום כן מספר הימים שיעמדו בשוה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דהיינו בי' ימים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כי אותו שמהלך בכל יום י' מילי' [126]מהלך בי' ימי' ק' מילי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואותו שמהלך ביום אחד א' מיל וביום שני ג' מילי' ומוסיף והולך על הדרך הזה ביום העשירי מהלך י"ט מילי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והרוב מי"ט הוא י' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תרבה י' בעצמם הוא ק' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Do this way for any number you want. | ועל זה הדרך תעשה לכל חשבון שתרצה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Word Problems - The Rule of Three |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| General Rule - A Selection of all the Rules in the World | ריגולא כללית והיא מבחר כל הריגולא שבעולם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Pricing Problems |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Find the Price | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם יאמ' אדם ג' ביצות שוי ד' פשיטים ה' ביצות לזה החשבון כמה פשיטים עולי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עשה על זה הדרך שתחזיר החשבון לאחורנית הוא שואל אם ג' שוה ד' ה' כמה שוה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואתה אמור ה' פעמים ד' חלקם בג' יהיו ו' וב' שלישיו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן אם שואל אם ה' שוה ז' ט' כמה שוי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור ט' פעמים ז' ס"ג חלקם בה' וכן יהיו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן אם יאמר י"א שוים י"ז ל"א לזה החשבון כמה שוים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור ל"א פעמים י"ז וחלקם בי"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| It is always this way. | וכן על זה הדרך לעולם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן אם יאמ' י' כורי' שוי' ו' דינרי' ד' כורי' לחשבון זה כמה שוי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור ד' פעמי' ו' כ"ד חלקם בי' יבואו ב' וד' עשיריות שהם ב' דינרי' וב' חומשי דינר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן אם יאמר ג' עמרים שוי ד' דינרי' י' עומרי' לחשבון זה כמה הם שוי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור י' פעמים ד' מ' חלקם בג' יהיו י"ג דינרי' ושליש דינר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Find the Amount | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור אם יאמר קניתי י' כורי חטה בו' דינרי' כמה כורי' אוכל ליקח בד' דינרי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור אם בו' דינרי' נמצא י' כורי' חטה בד' דינרי' כמה כורי' נמצא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור ד' פעמי' י' מ' חלקם בו' יהיו ו' וד' ששיות שהם ו' וב' שלישיות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן אם יאמר שש כורי' שוי' ד'[127] דינרי' כמה כורי אוכל לקנות בז' דינרי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור אם ד' דינרי' שוי' ו' כורי' ז' דינרי' כמה כורי' שוי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור ז' פעמים ו' מ"ב חלקם בד' יהיו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Employment Problems - Payment Problems |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The same for the issue of employment. | וכן לעניין השכירות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כגון האומר שכרתי פועל לל' יום בי' זוזים ועשה עמי ח' ימים כמה שכרו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור אם בל' יום יהיה שכרו י' זוז בח' ימים כמה שכרו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור ח' פעמים י' הם כזה פ' חלקם בל' יבואו ב' זוזי' וכ' חלקי' מל' שהם ב' וב' שלישיות שהם ג' דינרי' פחות שליש דינר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Calculation of Fractions |
חֶשְׁבּוֹן הַשְׁבָרִים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם תחלק האחד בב' חלקים יהיה בכל חלק מהם חצי ושני החצאי' הם אחד שלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן אם החלק האחד בג' חלקי' יהיה כל חלק מהם שליש ושלש השלישיות הם אחד שלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן אם תחלק האחד בד' חלקי' יהיה כל אחד מהם רביע וד' הרביעיי' הם אחד שלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| This way for all. | ועל זה הדרך הם כלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Addition of Fractions |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
![\scriptstyle{\color{OliveGreen}{\frac{1}{a}+\frac{1}{b}=\frac{\left[\frac{1}{a}\sdot\left(a\sdot b\right)\right]+\left[\frac{1}{b}\sdot\left(a\sdot b\right)\right]}{a\sdot b}}}](/mediawiki/images/math/2/6/4/264a022ee3bfdc1d622c23b20b6c5ffe.png)
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם יאמ' מי שיש לו החצי והשליש מדבר אחד כמה חלק יש לו מן הדבר ההוא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור חצי ושליש [128]ימצא בב' פעמים ג' שהם ו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חציי ג' ושלישיתו ב' ואם תקבצו יהיו ה' הרי שאותו שיש לו החצי והשליש מן הדבר יש לו ה' חלקי' מו' מן הדבר ההוא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם מי שיש לו השליש והרביעי מן הדבר כמה חלק יש לו ממנו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור שליש ורביע ימצא בג' פעמים ד' שהם י"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
השליש מהם הם ד' והרביעי הוא ג' ואם תקבצם הם ז' הרי שמי שיש לו השליש והרביע מן הדבר יש לו ז' חלקי' מי"ב מן הדבר ההוא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן מי שיש לו השליש והחומש מן הדבר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור שליש וחומש ימצא בג' פעמים ה' שהם ט"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
השליש מהם הוא ה' והחומש ג' ושניהם יחד הם ח' אם כן מי שיש לו השליש והחומש מן הדבר יש לו ח' חלקים מט"ו מן הדבר ההוא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן מי שיש לו הרביע והחומש מן הדבר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור רביע וחומש ימצא בד' פעמי' ה' שהם כ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הרביע הוא[129] ה' והחומש הוא ד'[130] ושניהם יחד הם ט'[131] אם כן יש לו ט' חלקים מכ' מן הדבר ההוא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן מי שיש לו שליש ושביע מן הדבר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור שליש ושביע ימצאו בג' פעמים ז' שהם כ"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
השליש ז' והשביעי ג' ושניהם יחד הם י' א"כ יש לו י'[132] חלקי' מכ"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן מי שיש לו שליש ורביע וחומש ושתות מן הדבר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור שליש ורביע וחומש ושתות אם ימצאו בס' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
השליש הוא כ' הרבי' ט"ו והחומש י"ב והשתות י' ואם תקבצם יהיו נ"ז הרי שיש לו נ"ז חלקים מס' מן הדבר ההוא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Subtraction of Fractions |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
![\scriptstyle{\color{OliveGreen}{\frac{1}{a}-\frac{1}{b}=\frac{\left[\frac{1}{a}\sdot\left(a\sdot b\right)\right]-\left[\frac{1}{b}\sdot\left(a\sdot b\right)\right]}{a\sdot b}}}](/mediawiki/images/math/c/0/6/c0629aecee310d90e051b57b2c593d46.png)
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמנם אם יאמר אדם כמה הוא יותר השליש מן הרביע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עשה על זה הדרך אמור שליש ורביע ימצאו בג' פעמי' ד' שהם י"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
השליש הוא ד' והרביעי הוא ג' כמה יותר ד' שהוא השליש מג' שהוא הרביעי א' א"כ השליש הוא יותר מן הרביע א' חלק מי"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן אם יאמר אדם כמה הוא יותר הרביע מן החומש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור רביע וחומש ימצאו בד' פעמי' ה' שהוא כ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הרביעי הוא ה' והחומש הוא ד' כמה הוא יותר ה' מד' א' אם כן הרביע הוא יותר מן החומש א' חלק מעשרים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן אם יאמר כמה הוא יותר השליש מן השביע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור שליש ושביע ימצאו בג' פעמים ז' שהם כ"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
השליש הוא ז' השביע הוא ג' כמה הוא יותר ז' על ג' ד' וא"כ השליש יותר מן השביע ד' חלקי' מכ"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם יאמר כמה הם יותר השליש והרביע מן החומש ושתות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור שליש ורביע וחומש ושתות ימצאו בס' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
השליש הוא כ' הרביע ט"ו אם תקבצם יהיו ל"ה והחומש מס' הוא ל"ב י"ב השתות י' אם תקבצם יהיו כ"ב כמה הם יותר ל"ה שהם השליש והרביע מכ"ב שהם החומש והשתות י"ג אם כן השליש והרביע הם יותר מן החומש והשתות י"ג חלקים מס' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Fractions of Fractions |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
![\scriptstyle{\color{OliveGreen}{\frac{1}{a}\sdot\frac{1}{b}=\frac{\frac{1}{a}\sdot\left[\frac{1}{b}\sdot\left(a\sdot b\right)\right]}{a\sdot b}}}](/mediawiki/images/math/6/a/0/6a0cf9b7b8a099931ff2b5d1e5906c1a.png)
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמנם אם יאמר מי שיש לו השליש מן הרביע מן הדבר כמה חלק יש לו ממנו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור שליש ורביע ימצא בי"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומי שיש לו הרביע מי"ב יש לו ג' חלקים מי"ב ומי שיש לו השליש מאלו הג' שהם הרביע יהיה לו א' חלק מי"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם יאמר מי שיש לו השליש מן החומש כמה חלק יש לו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור שליש וחומש ימצא בג' פעמים ה' שהם ט"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
החומש מט"ו הם ג' ומי שיש לו השליש מג' שהוא החומש יש לו א' חלק מט"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
[133]וכן מי שיש לו החומש מן התשיעית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור חומש ותשיע ימצא בה' פעמים ט' שהם מ"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והתשיעי ממ"ה הוא ה' והחומש מה' הוא א' א"כ מי שיש לו החומש מן התשיעית יש לו א' חלק ממ"ה מן הדבר ההוא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Conversion of fractions |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם יאמר אדם ג' שמיניות כמה עשיריות הם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עשה על זה הדרך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור שמינית ועשירי' ימצאו בח' פעמי' י' שהם פ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וג' שמיניות הם ל' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כי י' הוא שמינית פ'[134] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וח' הוא עשירית פ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תרצה לידע כמה עשיריות הם הג' שמיניות מפ' חלק הל' שהוא הג' שמיניות חלקם[135] בח' שהוא עשירית פ' הם ג' עשריות וו' ואותם הו' הם ו' חלקים מפ'[136] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Operations with fractions - numerator greater than 1 |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם יאמר הוסף ג' שמיניות על ז' עשיריות כמה יהיו[137] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור שמינית ועשירי' ימצאו בח' פעמים י' שהם פ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותוסיף ג' שמיניות והם ל' על ז' עשרותיו והם נ"ו יהיו הכל פ"ו חלקים מפ' שהם א' שלם וו' חלקים מפ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם יאמר השלך ג' שביעיות מב' שלישיות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמו' שלישית ושביעית ימצאו בג' פעמ' ז' שהם כ"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וקח ב' שלישיותיו והם י"ד ותשלך מהם ג' שביעיותיו והם ט' וישארו ה' חלקים מכ"א[138] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם יאמר כמה הם יותר ג' שמניות מב' חמשיות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמו' חמישיות ושמינית ימצא בה' פעמ' ח' שהם מ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ב' חמישיות הם י"ו וג' שמיניות הם ט"ו נמצא שהב' חמישיות הם יותר מג' שמיניות חלק אחת ממ'[139] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם יאמ' כמה הם ג' שמיניות מב' חמישיות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור שמינית וחמישית ימצאו בה' פע' ח' שהם מ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וב' חמישיות מ' הם י"ו וג' שמיניות י"ו הם ו' א"כ הם ו' חלקי' ממ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם יאמ' חלק ג' שמיניות על ב' חמשיות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמו' שמינית וחומש ימצאו בה' פעמ' ח' שהם מ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וג' שמניות מ' הם ט"ו וב' חמשיותיו הם י"ו חלק ט"ו על י"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם יאמר חשוב ב' שלישים עם ב' חמישיות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תחשוב שלשה על ה' שהם שמות החלקים יהיו ט"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואמור ב' פעמ' ב' כי ב' הם שמות החלקים ויהיו ד' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הרי שב' שלישיות על ב' חמישיות הם ד' חלקים מט"ו מן האחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם יאמר חשוב ב' חלקים מי"א באחד על ב' חלקים מי"ג מן האחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תחשוב שמות החלקים והם י"א על י"ג שעולים קמ"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותרבה מספר החלקים שהם ב' ואמור ב' פעמים ב' ד' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הרי שעולים ד' חלקים מקמ"ג בא' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם יאמ' ב' חלקים מה' בעשירית האחד בב' שלישי האחר כמה הם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דע כי שם האחד הם חמשים בעניין חמשה בעשרה ותמנה אלו הנ' בג' שהוא שם החלק השני ויהיה הכל ק"נ | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואמור ב' פעמ' ב' ד' כי ב' הוא מספר החלקי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הרי שהחשבו' הזה הוא ד' חלקי' מק"נ מהאחר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם יאמ' ב' חלקים מחמשה בעשירית האחד בשני חלקים משמנה בשלישית האחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אתה צריך לדעת את שמות החלקים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותחשוב ה' בי' והם נ' והוא שם החלק האחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותחשוב עוד ח' בג' ויהיו כ"ד והוא שם החלק השני | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וחשוב אחר כן נ' על כ"ד ויהיה אלף ומאתים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואמו' אחר כן ב' פעמ' ב' ר"ל ד' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הרי שהם ד' חלקים מאלף ומאתים מן האחר[140] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Multiplication of fractions |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כשנרבה השליש באחד הוא שליש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכשנרבה השליש בשנים הוא ב' שלישי האחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכשנרבה שליש בשליש הוא שליש בשליש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והרביע ברביע הוא רביע הרביע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וחומש בחומש הוא חומ' החומש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן כלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם נרבה ב' שלישי בב' שלישי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור ג' פעמים [.] ג' ט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואחר כך אמור ב' פעמים ב' פעמים הרי ד' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הרי שאם נרבה ב' שלישי על ב' שלישי הם ד' חלקי' מט' באחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Proportions with fractions |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם יאמר אדם אם שליש שוה רביע החומש כפי זה החשבון כמה שוה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור שליש ורביע וחומש ימצאו בס' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
השליש מס' הוא כ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
א"כ הרביע הוא ט"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והחומש הוא י"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואמור אם כ' שהוא שליש מס' שוה ט"ו שהוא הרביע החומש שהוא י"ב כמה שוה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור י"ב פעמי' ט"ו הם ק"פ חלקים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקם בכ' יבאו ט' והם ט' חלקים מס' באחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם יאמר אדם אם ג' ושליש שוים ד' ורביע ה' [ו]חומש כמה שוים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור ה' וחומש פעמים ד' ורביע וחלקם בג' ושליש וזה סדר עשייתו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תפוש ה' וחומש ואמור ה' פעמים ה' הם[141] כ"ה וא' חומש הרי כ"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אחר זה תפוש ד' ורביע ואמור ד' פעמים ד' י"ו וא' רביע הרי י"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותרבה י"ז עם כ"ו ויעלו תמ"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונחלק אותם בד' פעמים ה' שהם כ' יבואו כ"ב וא' עשירי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ובעבור שנצטרך לחלקם לג' ושליש עשה מכ"ב וא' עשירית כולם שלישיות ואמור ג"פ כ"ב ס"ו וג' פעמי' א' עשירית הרי ג' עשיריות הרי ס"ו וג' עשיריות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וצריך שנחלק אותם בג' ושליש וסדר חילופו שתעשה מג' ושליש כלם שלישיות ואמור ג'פ'ג' ט' וא' הרי י' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם נחלק ס"ו בי' חלקים יבואו ו' וו' עשיריות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נחלק אח"כ ג' עשיריות בי' חלקי' ישאר ג' חלקי' ממאה[142] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הרי שעולה החשבון ו' וו' עשיריות וג' חלקי' ממאה[143] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכדי שנוכל לקבץ הכל יחד ו' עשיריות חלקים ממאה ויהיו ס' חלקים מק' וג' חלקים מק' שהיו בידינו הרי ס"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תמצא מדברינו שאם ג' ושליש שוי' ד' ורביע ה' וחומש שוי' ו' וס"ג חלקים ממאה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Word Problems |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Exchange Problems |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם יאמר אדם ז' פיסאני שוים ט' קונטוניסי ק' ליט' פיסאני כמה ליט' קוטורניסי שוים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עשה על זה הדרך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ידוע כי אם ז' פיסאני שוים ט' קוטורניסי ז' דינ' פיסאני שוים ט' דנרי' קוטורניסי וז' ליט' פיסאני שוים ט' ליט' קוטורניסי ות"ש ליט' פיסאני שוים תת"ק ליט' קוטורניסי וכן לכל מטבע שתרצה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
א"כ השאלה הזאת היא כאלו שאל אם ת"ש ליט' פיסאני שוים תת"ק ליט' קוטורניסי ק' ליט' פיסאני כמה שוים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולהקל החשבון נאמר אם ז' שוים ט' ק' כמה שוים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור ק' פעמי' ט' תת"ק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקם בז' יהיו קכ"ח ליט' וי"א דינ' וא' שביע וכן ישוו הק' ליט' פיסא' לקוטורניסי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם יאמר אדם אם ה' פיסאני ורביע שוים ז' קוטורניסי אלף ליט' פיסאני כמה ליט מקוטורניסי שוים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנך רואה כי זה החשבון הוא שבור מחלק אחד וזה השבור הוא רביע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
לכן נרבה הב' חלקים שהם ה' ורביע וז' בד' דהיינו שנעשה כלם רביעיים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואמ' ד' פעמי' ה' פיסאני ורביע יבואו כ"א פיסאני | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וד' פעמי' ז' קוטורניסי יבואו כ"ח קוטורניסי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הרי שכ"א פיסאני שוים כ"ח קוטורניסי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולהקל הענין נחלקם בשביע בעבור שבשני החלקים ימצא שביע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
השביע מכ"א הוא ג' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והשביע מכ"ח הוא ד' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נמצא שג' פיסאני שוים ד' קוטורניסי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואנו רוצים לדעת כמה שוים אלף ליט' פיסאני | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור א"כ אם ג' פיסאני שוים ד' קוטורניסי אלף ליט' פיסאני כמה שוים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור אלף פעמי' ד' יבואו ד' אלפים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקם בג' יבואו אלף ושל"ג וו' די' וח' פשוטי' וכן שוים אלף ליט' פיסאני לקוטרניסי[144] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
[145]ואם יאמר אדם אם ז' אונקיו' ושליש מכסף שוי' ע"ג ליטרי' ורביע י"ט אונקיו' מכסף כמה ישוו לפי חשבון זה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עשה על זה הדרך השברי' שהם בזה החשבון הם שליש ורביע ושליש ורביע ימצאו בי"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
לכן יש לנו לרבות הב' חלקי' שהם ז' ושליש וע"ג ורביע בי"ב דהיינו לעשות מכלם חלקים מי"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואמור י"ב פעמי' ז' ושליש יבואו פ"ח אונקיו' כסף | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וי"ב פעמים ע"ג ליטרי' וה' דינרי' יהיו ת"תע"ט ליט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הרי שפ"ח אונקיו' כסף שוי' תתע"ט ליטרי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואנו רוצי' לידע כמה שוי' הי"ט אונקיו' לחשבון זה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור אם כן אם פ"ח אונקיו' כסף שוי' תתע"ט ליטרי' ואנו רוצי' לידע כמה שוי הי"ט אונקיו' לחשבון זה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור י"ט פעמי' תתע"ט יהיו י"ו אלפים ותש"א ליט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלק אותם בפ"ח דהיינו בח' ובי"א יבואו קפ"ט ליטרי' וט"ו דינרי' וח' פשיטי' וב' חלקי' מי"א מהפשוט וכן ישוו הי"ט אונקיו' כסף לפי חשבון זה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Divide a Quantity Problems |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם תרצה לחלק ה' פשיטים לשליש ולרביע ולא ישארו מהם כלל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור שליש ורביע ימצא בג' פעמים ד' שהם י"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
השליש הוא ד' הרביע הוא ג' וכללם הוא ז' והוא המחלק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם יאמר תרצה לדעת כמה הוא חלק אותו שיש לו השליש אמור ד' פעמי' ה' בעבור שהם ה' יבואו כ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תרצה לדעת חלק אותו שיש לו הרביע אמור ג' פעמי' ה' ט"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תקבץ ב' וו' חלקים מז' וב' וא' חלק מז' יהיו ה' פשיטי' כמספר המעות שחלקת | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תרצה לחלק י"ב פשי' לחצי לשליש ולרביע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור חצי ושליש ורביע ימצאו בי"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
החצי הוא ו' השליש הוא ד' הרביע ג' וכללם י"ג והוא המחלק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תרצה לדעת חלק מי שיש לו החצי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור החצי מי"ב הוא ו' תרבה אותם עם י"ב שהוא סך המעות יהיו ע"ב חלקם בי"ג יהיו ה' וז' חלקים מי"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תרצה לדעת חלק אותו שיש לו השליש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור השליש מי"ב הוא ד' ותרבה אותם עם סך המעות שהם י"ב ויהיו מ"ח וחלקם בי"ג יהיו ג' וט' חלקים מי"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תרצה לדעת חלק אותו שיש לו הרביע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור הרביע מי"ב הוא ג' תרבה אותו עם סך המעות שהם י"ב ויהיו ל"ו חלקם בי"ג יהיו ב' וי' חלקי' מי"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תקבץ ג' החלקים האלה יהיו י"ב כמספר המעו' שחלקת | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן אם תרצה לחלק י"ב פשיטי' לשליש ולרביע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור שליש ורבי' ימצאו א בי"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
השליש הוא ד' והרביע הוא ג' וכללם ז' והוא המחלק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תרצה לדעת מי שיש לו השליש כמה יהיו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור שליש מי"ב הם ד' תרבה אותם על סך המעות שיהיו י"ב ויהיו מ"ח חלקם בז' יהיו ו' וו' חלקי' מז' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולדעת אותו שיש לו הרביע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור הרביע מי"ב הוא ג' תרבה [146]אותם עם י"ב יהיה הרביע ל"ו חלקם בז' יהיו ה' וא' חלק מז' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ושניהם י"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם יהיו בידך ל' פשיטי' ותרצה לחלק אותם לשליש ולרביע ולחומש ולשתות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור החלקי' האלה ימצאו בס' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
השליש הוא כ' הרביע ט"ו החומש י"ב השתות י' וכלם נ"ז והוא המחלק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואותו שיש לו השליש יאמר השליש מס' הוא כ' ותרבה אותם עם ל' שהוא סך המעות יהיו ת"ר חלקי' בנ"ז יבואו י' ול' חלקי' מנ"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואותו שיש לו הרביע שהוא ט"ו יאמר ט"ו פעמי' ל' הם ת"ן חלקם בנ"ז יהיו ז' ונ"א חלקי' מנ"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואותו שיש לו החומש מי"ב שהוא י"ב יאמר י"ב פעמי' ל' הם ש"ס חלקם בנ"ז יבאו ו' וי"ח חלקי' מנ"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואותו שיש לו השתות שהוא י' יאמר י' פעמי' ל' הם ש"ס ש' חלקם בנ"ז יהיו ה' וט"ו חלקי' מנ"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכלל כל החלקי' הנזכרי' הם ל' פשיטי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
לחלק ס' ליטרי' לחצי לשליש ולרביע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור חצי ושליש ורביע ימצאו בי"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכללם י"ג והוא המחלק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תרצה לדעת חלק אותו שיש לו החצי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור החצי מי"ב הוא ו' תרבה אות' עם ס' שהוא סכום ה' ליטרי' יהיו ש"ס חלקם בי"ג יהיו כ"ז וט' חלקים מי"ג מהליטר' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תרצה לדעת חלק אותו שיש לו השליש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור השליש מי"ב הם ד' תרבה אותם עם ס' יהיו ר"מ חלקם בי"ג יהיו יהיו י"ח וו' חלקי' מי"ג מן הליטר' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תרצה לדעת אותו שיש לו הרביע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור הרביע מי"ב הוא ג' תרבה אותם עם ס' יהיו ק"פ חלקם בי"ג הוא י"ג וי"א חלקי' מי"ג מן הליט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וא' חלק מי"ג מן הליטר' הוא י"ח פשיטי' וב' חלקי' מי"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
יהיה אם כן חלק אותו שיש לו החצי כ"ז ליטרי' וי"ג דינרי' וי' פשיטי' וב' חלקי' מי"ג מן הפשוט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וחלק אותו שיש לו השליש הוא י"ח ליטרי' וט' דינרי' וב' פשיטי' וי' חלקי' מי"ג מן הפשוט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וחלק אותו שיש לו הרביע הוא י"ג ליטרי' וי"ו דינרי' וי"א פשיטי' וא' חלק מי"ג מן הפשוט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תקבץ כל החלקים הנזכרי' יהיו ס' ליטרי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Partnership - for the same time |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שלשה אנשים עשו חברותה ושמו בין שלשתם א' אונקי' זהב לאחד היה זהב שוה ג' ליטרי' האונקי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור שליש וחומש ושמין ימצאו בק"כ | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
השליש הוא מ' החומש הוא כ"ד השמינית ט"ו וכללם ע"ט והוא המחלק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תרצה לדעת כמה זהב ישים אותו שהזהב שלו שוה ג' ליטרי' האונקי' אמור השליש מק"כ הוא מ' ותרבה אותו עם סך האונקיות [147]שהם מ' יהיו אלף ות"ר[148] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תרצה לדעת כמה שוה חלקו אמור הכ' אונקיו' לחשבון ג' לי' האונ' עולי' ס' ליטרי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומן הכ' חלקי' מע"ט אמור אם ע"ט שהוא כ' ליטרי' א' אונקי' שוה תש"כ פשוטי' שהם ג' ליט' כ' חלקים מע"ט כמה שווים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמו' כ' פעמ' תש"כ[149] יהיו חלקם בע"ט יבואו קפ"ב פשיטי' וכ"ב חלקים מע"ט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שהם ס' ליט'[150] וט"ו דינרי' וב"פ וכ"ב חלקים מע"ט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תרצה לדעת כמה זהב ישים אותו שהזהב שלו שוה ה' ליטרי' האונ' אמור החומש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תרצה לדעת כמה שוה חלקו אמור הי"ב אונקיו' לחשבון ה' ליטרי' האונקי' עולי' ס' ליטרי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומן הי"ב חלקים[152] מע"ט [אמור אם ע"ט שהוא א' אונקי' עולה אלף ור' פשוטי' שהם ה' ליט' הי"ב חלקים מע"ט כמה שווים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמו' י"ב פעמ' אלף ור' הם י"ד אלפים ות' חלקם בע"ט יבואו קפ"ב פשוטי' וכ"ב חלקים מע"ט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הרי ששווה חלקו ס' ליט' וט"ו די' וב"פ וכ"ב חלקים מע"ט כמו הראשון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תרצה לדעת כמה זהב ישים אותו שהזהב שלו שווה ח' ליט' האונק' אמו' השמינית מק"כ הם ט"ו תרבה אותם עם מ' שהם סכום האונק' יהיו ת"ר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תרצה לדעת כמה שוה חלקו שלזה אמו' הז' אונק' לחשבו' ח' ליט' עולים נ"ו ליט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומן המ"ז חלקים מע"ט אמו' אם ע"ט שהוא א' אונק' שוה אלף ותתק"כ פשוט' שהוא ח' ליט' המ"ז חלקים מע"ט כמה שווים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמו' מ"ז פעמ' אלף ותתק"כ הם צ' אלפים ור"מ חלקם בע"ט יהיו אלף וקמ"ב פשוטי' וכ"ב חלקים מע"ט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שהם ד' ליט' וט"ו די' וב"פ וכ"ב חלקים מע"ט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
צרף אותם עם הנ"ו ליט' הרי ששווה חלקו שלזה כחלק כל אחד מחבריו ס' ליט' וט"ו די' וב"פ וכ"ב חלקים מע"ט][153] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Multiple Quantities Problems |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שלשה אחים לשיני יש יותר מן הראשו' על אחת ג' ולשלישי יש לאחת על אחת שיני ג' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עשה לך מורה שיוסיף לך זה על זה כזה הדמיון והם א' ג' ט' ומחובר שלשתם י"ג יהיה לראשון א' ולשני ג' ולשלישי ט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועתה שאמר ומחובר שלשתם ס'[154] עשה על זה הדרך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולדעת כמה יש לראשון אמור אם בהיות המחובר י"ג יהיה החלק הראשון א' עתה שהמחובר ס' כמה יש לו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור ס' פעמי' א' הם ס' חלקם בי"ג יהיו ד' וח'[155] חלקים מי"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולדעת חלק השיני אמור אם בהיות המחובר י"ג יהיה החלק[156] השני ג'[157] עתה שהמחובר ס' כמה הוא חלקו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמו' ס' פעמי' ג' הם ק"פ חלקם בי"ג יהיו י"ג וי"א חלקים מי"ג[158] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולדעת חלק השלישי אמור אם בהיות המחובר י"ג היה חלק השלישי ט' עתה בהיות המחובר ס' כמה הוא חלקו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור ס' פעמי' ט' הם תק"מ חלקם בי"ג יהיו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תקבצם יהיו כלם ס' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן אם יהיו זה על זה ב' או ד' או ה' וכן אם יהיו יותר מג' חברים כמו כן עשה המורה על דרך הראוי ותמצא חפצך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
יעקב חלק לחמשה בניו ל"ה דינרים והיה חלק ראובן גדול משל לוי ושל לוי גדול משל יששכר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה [159]לפי זה חלק ראובן ט"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וחלק לוי ה' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וחלק יששכר ג' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וחלק שמעון י' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וחלק יהודה ב' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכך כלם ל"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה אמר השואל יעקב חלק לה' בניו ס' דינרי' כפי השאלה הקודמ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עשה כפי זה הדרך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם תרצה לדעת חלק ראובן אמור אם בהיות סכום המעות ל"ה היה חלק ראובן ט"ו בהיות סכום[160] הדינרי' ס' כמה הוא חלקו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור ס' פעמים ט"ו[161] הם תת"ק חלקם בל"ה יהיו כ"ה וכ"ה חלקים מל"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולדעת חלק לוי אמור אם בהיות סכום הדינרי' ל"ה היה חלק לוי ה' בהיות סכום המעות ח' כמה יהיו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור ס' פעמי' ה'[162] הם ש' חלקם בל"ה יהיו ח' דינרי' וכ' חלקים מל"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולדעת חלק יששכר אמור אם בהיות סך המעות ל"ה דינרי' היה חלקו ג'[163] בהיות סך המעות ס' כמה יהיו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור ס' פעמים ג' הם ק"פ חלקם בל"ה יהיו ה' וה' חלקים מל"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולדעת חלק [שמעון שהוא כעודף ראובן על לוי יהיה חלקו י"ז וה' חלקים מל"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולדעת חלק][164] יהודה שהוא כעודף לוי על יששכר יהיו חלקו ג' וט"ו חלקי' מל"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תקבץ כל המספרים יהיו ס' ותמצ' [חלק][165] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Joint Purchase Problem - If You Give Me - Amounts of Money |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שלשה חברים אומר הראשון לשני כל מה שבכיסי וחצי שניכם עולה ס' ענה השיני כל מה שבכיסי ושליש שניכם עולה ס' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
מצאנו ג' מספרי' והם ה' י"א י"ג שהם כעין השאילה הזאת | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כי ה' אומ' לי"א ולי"ג אני וחצי שניכם י"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וי"א אומ' לי"ג [וה'][166] אני ושליש שניכם י"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
י"ג אומרת לי"א ולה' אני ורביע שניכם י"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנך רואה שאמרו מחובר שלשתינו י"ז יהיה לאחד ה' ולשני י"א ולשלישי י"ג ועכשיו שאמרו מחובר שלשתינו ס' נשיב לערכים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונאמר כערך ה' לי"ז כן ערך ראשון לס' הוא ששאל לחביריו החצי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכערך י"א לי"ז כן ערך האמצעי [לס'][167] הוא ששאל לחבירו השליש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכערך י"ג לי"ז כן ערך הגדול אל ס' והוא ששאל לחביריו הרביע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן תעריך לכל הסכומים שיאמרו שלשתם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| תשיב המורה ה' י"א י"ג | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ודרך הערכים הוא בעניין הזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
[אם תרצה לדעת חלק הקטון אמור אם בעת שהמחובר י"ז יהיה חלק הקטן ה' בהיות המחובר ס' כמה הם חלקו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור ס' פעמי' ה' הם ש' חלקם בי"ז יהיו י"ז וי"א חלקים מי"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולדעת חלק האמצעי אמור אם בעת שהמחבר י"ז שוה החלק האמצעי י"א בהיות המחבר ס' כמה הוא חלקו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור ס' פעמי' י"א הם תר"ס חלקם בי"ז יהיו ל"ח וי"ד חלקים מי"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולדעת חלק הגדול אמור אם בעת שהמחבר הוא י"ז יהיה חלק הגדול י"ג בהיות המחובר ס' כמה הוא חלקו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור ס' פעמי' י"ג הם תש"פ חלקם בי"ז יהיו מ"ה וט"ו חלקים מי"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ויהיה סדר העניין כן | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אומר הקטן אני שאני י"ז וי"א חלקים מי"ז וחצי האמצעי שהם י"ט וז' חלקים מי"ז וחצי הגדול שהם כ"ב וי"ו חלקים מי"ז מחובר שלשתינו עולה ס' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואומר האמצעי אני שאני ל"ח וי"ד חלקים מי"ז ושליש הקטן שהם ה' וט"ו חלקים מי"ז ושליש הגדול שהם ט"ו וה' חלקים מי"ז מחובר שלשתינו עולה שהם ס' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואמר הגדול אני שאני מ"ה וט"ו חלקים מי"ז רביע הקטן שהוא ד' וז' חלקים מי"ז ורביע האמצעי שהוא ט' וי"ב חלקים מי"ז מחובר שלשתינו עולה ס'][168] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Multiple Quantities Problems |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Three Brothers - Money |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שאלה יעקב חלק [כ"ד][169] דינרים לג' בניו והיה חלק ראובן גדול משל שמעון וחלק שמעון גדול מחלק לוי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור כי החלק האחד היה ד' והחלק השני ז' והחלק השלישי י"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונמצא באחרונה חלק כל אחד מהם ח' שוה בשוה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והיה חלק ראובן י"ג וחלק שמעו' ז' וחלק לוי ד' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה התחיל ראובן ונתן מן הי"ג שלו ז' לשמעון כפי חלקו וד' [ל]לוי כפי חלקו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
[ושמעון שחלקו מעתה הם י"ד נתן ב' לראובן כפי חלקו וח' ללוי כפי חלקו][170] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולוי שחלקו הם מעתה י"ו נתן ד' לראובן וד' לשמעון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
מעתה יש לכל אחד ואחד מהם ח' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Selling Cloth |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אדם אחד נתן לשלש[ת] [בניו][171] [172]שלשי' אמות בגד למכור הלכו בשוק למכרו האחד מכר בד' דינרי' האמה והשני מכר בה' דינרי' האמה והשלישי מכר בו' דינרי' האמה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור תחלה רביע וחומש ושתות ימצאו בס' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הרביע הוא ט"ו והחומש הוא י"ב והשתות י' וכללם ל"ז והוא המחלק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תרצה לדעת כמה בגד מכר אותו שמכר בד' דינרי' האמה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור הרביע מט"ו מס' הוא ט"ו ותרבה אותו על סך הבגד שהו' ל' אמות ואמור ט"ו פעמים ל' הם ת"נ חלקם בל"ז שהוא המחלק יבאו י"ב אמות וו' חלקי' מל"ז מן האמה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תרצה לדעת כמה מעות קבל זה שמכר בד' דינרי' האמה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור מי"ב אמות קבל מ"ח דינרי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומן הו' חלקים מל"ז עשה על זה הדרך אמור אם ל"ז שהם אמה שלמה שוה מ"ח פשיטי' שהם ד' דינרי' הו' חלקי' מל"ז כמה שוי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור ו' פעמי' מ"ח הם רפ"ח חלקם בל"ז יבואו ז"פ וכ"ט חלקים מל"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הרי שמכר י"ב אמות וו' חלקי' מל"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וקיבל מ"ח דינרי' וז"פ וכ"ט חלקי' מל"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תרצה לדעת כמה בגד מכר אותו שמכר בה' דינרי' האמה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור החומש מס' הוא י"ב תרבה אותם עם סכום הבגד שהם ל' אמות ואמור י"ב פעמים ל' והם ש"ס חלקם בל"ז שהוא המחלק יבואו ט' אמות וכ"ז חלקים מל"ז מן האמה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תרצה לדעת כמה מעות קבל אותו שמכר בה' דינרי' האמה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור מט' אמות [בה' די' האמה][173] קבל מ"ה דינרי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומן ה"ז הכ"ז חלקים מל"ז עשה על דרך הראשון ואמור אם מל"ז שהוא א' שלם שוי' ס' פשיטי' שהם ה' דינרי' הכ"ז חלקי' מל"ז כמה הם שוי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור כ"ז פעמי' ס' הם אלף ותר"כ חלקם בל"ז יבואו מ"ג פשיטים וכ"ט חלקי' מל"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
[וצרף אותם עם המ"ה די' שקבל מט' אמות בגד שמכר הרי שזה שמכר בה' די' האמה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומכר ט' אמו' וכ"ז חלקים מל"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
קבל מ"ח די' וז' פשו' וכ"ט חלקים מל"ז][174] כמו הראשון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תרצה לדעת כמה בגד מכר אותו שמכר בו' דינרי' האמה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור [השתות מס' הוא י' תרבה אותם עם סכום הבגד שהם ל' אמות ואמו' י' פעמ' ל' הם ש' חלקם בל"ז יהיו ח' אמות וד' חלקים מל"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תרצה לדעת כמה מעות קבל זה שמכר בו' די' האמה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמו'][175] מן הח' אמות קבל מ"ח דינרי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומן הד' חלקים מל"ז אמור אם ל"ז שהוא אחד שלם שוה ע"ב פשיטי' שהם ו' דינרי' הד' חלקי' מל"ז כמה הם שוי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור ד' פעמים ע"ב הוא רפ"ח חלקם בל"ז יהיו ז"פ וכ"ט חלקים מל"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הרי שזה שמכר בו' דינרי' האמה מכר ח' אמות וד' חלקי' מל"ז אמות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וקבל מ"ח וז"פ וכ"ט חלקי' מל"ז ככל אחד מחבירו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Mixture and Alligation Problem |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הרי שנתת לצורף י' אונקיות זהב לעשות ממנו מעות הב' אונקיות מהם היה זהב מי"ד קראטי האונקי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואתה קח סכום כל הא' [176]על האוקיו' של זהב וקח סכום הקראטי ג"כ וחלק הקראטי לסכום האוקיו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כיצד הב' אוקיו' לי"ד קראטי האוקי' עולות כ"ח קראטי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והג' אונקיו' מי"ו קראטי האוקיו' עולי' מ"ח קראטי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והה' אונקיו' מי"ח קראטי עולות צ' קאראטי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עולי' קס"ו קראטי בין הכל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקם בעשרות כמניין האוקיו' יבא לכל אונקי' י"ו קראטי וג' חומשי קראטי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן לכל חשבון שתרצה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Pricing Problems |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כשתדע שהזהב הוא מי"ח קראטי האונקי' ותרצה לדעת כמה פרחי' שוה האוקי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עשה מכל י"ח קראטי שלישיות וי"ח שלישיות הם ו' שלמים הרי שהזהב שהוא מי"ח קראטי שוה האונקי' ממנו ו' פרחי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמת שאתה צרי' לפחות מכל קראטי ו' פשי' בעבור שזה הזהב אינו קוניינטו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם הזהב מעשרי' קראטי ותרצה לדע' כמה שוה האונקי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עשה מן הכ' קראטי שלישיות הרי שהזהב שהוא מכ' קראטי שוה אונקי' ממנו ו' פרחי' וב' שלישי פרח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואתה צרי' לעולם לפחות ו"פ לכל קראטו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן אם הזהב הוא מכ"א קראטי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור כ"א שלישיות הם ז' שלימים הרי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן אם הזהב הוא מכ"ד קראטי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור כ"ד שלישיות הם ח' שלימים הרי שהאוקי' שוה ח' פרחי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואתה צריך לפחות ו' פשיטי לכל קראטו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שיבואו י"ב דינרי' בין כל האונקיו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והאונקי' שוקלת ח' פרחי' שיבואו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הרי שהזהב מפרח שאינו קוניינטו שוה י"ח פ' פחו' מא' פרח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ארבע נהרות רצים אל מעיין אחד האחד ממלאו ביום אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עשה על זה הדרך אמור ראשון ושליש ורביע ימצאו י"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הראשון הוא י"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
החצי ו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
השליש ד' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הרביעי ג' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חבר אותם יחד יהיו כ"ה והוא המחלק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכשתתבונן תמצא שי"ב ימים ימלאו כ"ה מעיינות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כיצד הממלאו ביום אחד ביום בי"ב ימים ימלא י"ב מעיינות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והממלאו בב' ימים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והממלאו בג' ימים בי"ב ימים ימלא [ד'][178] מעיינות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והממלאו בד' ימים בי"ב ימים ימלא ג' מעיינות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הרי שארבעתם יחד ימלאו בי"ב ימים כ"ה מעיינות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור אם כן כ"ה מעיינות יתמלאו בי"ב ימים א' מעיין בכמה חלק מן היום יתמלא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור א' פעם י"ב י"ב חלקם בכ"ה יבואו י"ב חלקים מכ"ה הרי שי"ב חלקים מכ"ה [179]מהיום ממלאים אותו כשירוצו מארבעתם יחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תרצה לדעת כמה חלק מן המים שם כל אחד מן הנהרות במעיין | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור הראשון הוא י"ב וי"ב פעמים א' בעבור המעיין שהוא א' הרי י"ב חלקם בכ"ה יבואו י"ב חלקים מכ"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואותו הממלאו בב' ימים אמור החצי מי"ב הוא ו' אמור ו' פעמי' א' בעבור המעיין הם ו' חלקם בכ"ה יבואו ו' חלקים מכ"ה הרי ששם במעיין ו' חלקים מכ"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והממלאו בג' ימים אמור השליש מי"ב הוא ד' אמו' ד' פעמי' א' ד' חלקם בכ"ה יבואו ד' חלקים מכ"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והממלאו בד' ימים אמור הרביע מי"ב הוא ג' אמור ג' פעמים א' ג' חלקם בכ"ה הרי ששם במעיין ג' חלקי' מכ"ה מן המים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועל זה הדרך תעשה לכל חשבון שתרצה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Multiple Quantities Problems |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אדם אחד היה לו בנים לא נדע מספרם והיו לו פרחי' לא אומר לך כמה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עשה על זה הדרך בעבור שהחשבון השבור שבזה החשבון הוא עשירית קח דוגמתו עשרה ותפחות מהם אחד ישארו ט' וט' היו הבנים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וט' פעמים ט' היו הפרחים והם פ"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וט' פרחים היה חלק כל אחד ואחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כיצד הראשון קבל א' פרח מן הפ"א ועשירית הנשאר שהוא ח' הרי שקיבל ט' פרחי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונשארו ע"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
השני קבל ב' פרחים מן הע"ב ועשירית הנשאר שהם ז' הרי שקיבל ט' פרחי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונשארו ס"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
השלישי קיבל ג' פרחי' מן הס"ג ועשירית הנשאר שהם ו' הרי שקבל ט' פרחי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונשארו נ"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הרביעי קבל ד' מן הנ"ד ועשירית הנשאר שהם ה' פרחי' הרי שקבל ט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונשארו מ"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
החמשי קבל ה' פרחי' מן המ"ה ועשירית מן הנשאר שהם ד' הרי שקיבל ט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונשארו ל"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הששי קבל ו' פרחי' א מן הל"ו ועשירית מן הנשאר שהם ג' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונשארו כ"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
השביעי קיבל ז' פרחי' מן הכ"ז ועשירית הכ' הנשארי' שהם ב' הרי שקבל ט' פרחי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונשארו י"ח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
השמיני לקח ח' פר' מן הי"ח ועשירית הי' הנשארים שהוא א' הרי שקבל ט' פרחי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונשארו ט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
התשיעי שהוא האחרון הלך ולקח הכל שהם הט' פרחי' הנשארי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הרי שט' היו הבני' וט' פע' ט' היו הפרחי' וט' פרחי' היה חלק כל אחד ואחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
[180]ודע כי זאת הריגולא היא כללם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכמו כן אם אמר לראשון קח א' פרח ותשיעית הנשאר ולשני ב' ותשיעית הנשאר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
יעשה על דרך הנזכר דהיינו בעבור שהחשבון השבור בזה החשבון הוא תשיעית יקח דוגמתו תשעה ויפחות מהם אחד וישארו שמנה ושמנה היו הבנים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וח' פע' ח' שהם ס"ד וס"ד היו הפרחים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וח' פרחי' יבואו לכל אחד ואחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן לכל חשבון שתרצה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Rent Problems |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם יהיה שכירות הבית ו' ליטרין לשנה או אם יהיה לך חשבון שירויח השנה ו' ליטרי' ותרצה לידע כמה יבא היום | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תפוש הב' שלישיות מו' שהם ד' וכן יבואו אילו ד"פ ליום אחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן אם יהיה שכירות הבית או ריוח החשבון ט' ליטרי' השנה ותרצה לדעת כמה יבא היום | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
קח מב' חלקים מט' שהם ו' יבואו ו"פ ליום | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן יהיה שכירות הבית או ריוח החשבון י"ב ליטרי' השנה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
קח שני שלשיות והם ח' וכן יבואו ח' פשיטים בכל יום | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Or, do this way: double the [number of] liṭra earned a year, then divide them into a third; the result are the pešiṭim earned a day.
|
או תעשה בעניין זה תכפול הליטר' שיבואו השנה וחלקם לשליש וכמספר העולה יהיו הפשיטי' שיבואו ליום | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה אם יהיה השכירו ו' ליטרין השנה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תכפלם יהיו י"ב חלקם לשליש יהיו ד' הרי שיבואו ד"פ ליום | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן אם יהיה השכירות ט' ליטרי' השנה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפלם יהיו י"ח חלקם לשליש יהיו ו' הרי שיבואו ו' פשיטי' ליום | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן אם יהיה השכירות י"ב ליט' השנה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפלם יהיו כ"ד חלקם לשליש יהיו [ח'][181] וכן יהיו ח"פ ליום | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועל זה הדרך תעשה לכל חשבון הדומה לזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם יאמר אדם [שכירות הבית או ריוח חשבון אחד עולה ד"פ ליום כמה ליט' יבואו השנה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הוסף על ד' חציים שהם ב' ויהיו ו' וכן יבואו לחשבון זה ו' ליטרי' [השכיר'][182] השנה][183] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
[184]וכן אם יאמר שכירות הבית ו' ליטרי' בשנה ו' פשיטי' ליום כמה ליטרי' יבואו השנה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הוסף על ו' חציים ג' הוא ט' וכן יבא ט' לשנה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן אם יאמר שכירות הבית הוא ח' פשיטים ליום כמה ליטרי' יבאו השנה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הוסף על ח' חציים שהם ד' יהיו י"ב וכך יהיו י"ב ליטרי' לשנ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועל זה הדרך תעשה כל חשבון שתרצה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Pricing Problems |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
[185]אם תקנה הככר מן הלוחות או מאיזה דבר שתרצה בז' ליט' ותרצה לדעת כמה יבא האחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
קח לכל ליט' ב' פשוטי' וב' חמישייו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם כן תכפול ז' ויהיו י"ד פשוטי' וי"ד חומשים הרי בן כולם י"ז פשוטי' פחו' מחומש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן אם תקנה הככר בה' ליט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפול ה' יהיו י' הרי שיבוא לזה החשבו' י' פשוטי' וי' חמשייו' שהם י"ב פשוטים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן אם תקנה הככר בט' ליט' ליט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפול ט' יהיו יהיו י"ח וי"ח חמשיות שהם כ"א פשו' וג' חמשיות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן לחשבון כ"ה ליט' הככר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
יבא האחד נ' פשו' ונ' חמשיות שהם ה' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן לחשבון ל' ליט' הככר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
יבא האחד ס' פשו' וס' חמשיות שהם ו' דינ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם יהיו שם די' כגון שיבא הככר י' ליט' וג' די' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
קח לכל די' ג' חלקים מכ"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומן הי' ליט' יבואו ב' די' כמו שידעת | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ובעבו' הג' די' יבואו ט' חלקים מכ"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן לעולם תוסיף לכל די' ג' חלקים מכ"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Interest and Discount Problems - Find the Earned Interest |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
[ואם תתן י"ב ליט' מן הככר לשנה ותרצה לידע לחשבו' כמה פשוטי' הליט' יבוא החודש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תפוש מכל ליט' שיבא הככר א' חומש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם כן יבא לחשבו' י"ב חומשי' דהיינו לחשבו' ב' פשוטי' וב' חמישייו' החודש הליט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולחשבו' ז' ליט' הככר לשנה יבוא הליט' א' חודש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
לחשבו' ז' חומשים שהוא א' פשוט וב' חמישייו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולחשבון ח' ליט' הככר לשנה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
יבא חמשים שהם א' פשוט וג' חמשי' פשוטי' לליט' בחדש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולחש[ב]ון י' ליט' הככר לשנה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
יבא לחשבון י' חמשים שהוא לחשבון ב' פשו' החדש לליט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן לכל חשבון שתרצה][186] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם תלוה שלשה דינרי' לחשבון ו' פשיטי' החדש הליטר' ותרצה לידע כמה יבא החדש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור אם מכ' דינרים יבא ו' פשיטי' מג' דינרי' כמה יעלו יבאו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור ג"פ ו' י"ח חלקם בכ' יבואו י"ח חלקים מכ' בפשוט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
[וכן אם תלוה ה' דינרי' לחשבון][187] ז' פשיטי' הלי' השנה ותרצה לידע כמה יבא בחדש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור אם מכ' דינרי' יבא ז' פשי' מה' דינרי' כמה יבואו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור ה' פעמים ז' ל"ה חלקם בכ' יבואו א' פשוט וט"ו חלקים מכ' בפשוט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועל זה הדרך תעשה לעולם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם תלוה שלשה דינרי' לחשבון [ו' פשו' הליט' בחדש][188] ועמדו ט' חדשי' ותרצה לידע כמה יבואו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור ג' פעמים [ט'][189] הם כ"ז וכמו שיבואו כ"ז דינרי' א' חודש לחשבון ול ו"פ הלי' השנה כן יבאו ג' דינרי' ט' חדשים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן אם תלוה ט ז' דינרי' לחשבון ה' פשיטי' הלי' השנה ועמדו יד י' חדשים ותרצה לדעת כמה יבאו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור ז' פעמי' י' הם ע' וכמו שיבאו ע' דינרי' שהם ג' ליטרין וחצי א' חדש הליט' לחשבון ה' פשיטי' הליט' השנה כן יבואו הז' דינרי' י' חדשים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן אם תלוה ט' דינרי' לחשבון ה' פשי' הליט' השנה ועמדו ה' חדשים ותרצה לדעת כמה יבואו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור ה' פעמי' ט' מ"ה וכמו שיבואו מ"ה דינרי' א' חדש לחשבון ד"פ הלי' השנה כן יבואו הט' דינרי' ה' חדשים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן לכל חשבון שתרצה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם תלוה הככר בי"ב ליטרי' [190]השנה ותרצה לידע כמה יבא החדש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דרך ריגולא תרבה הליט' שיבואו השנה לעולם על ה' ומה שיעלה יהיה ידות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה אמור י"ב פעמים ה' הם ס' נמצא שהם ס' ידות שהם כ' דינרי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תלוה הכיכר לחשבון ה' ליטרי' לשנה ותרצה לידע כמה יבא החדש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור ה' פעמים [ה'][191] כ"ה והם כ"ה ידות שהם ח' דינרי' וד' פשיטי' וכן יבא החדש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן אם תלוה הכיכר לחשבון ו' ליטרי' השנה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור ה' פעמים ו' הם ל' והם ל' ידות שהם י' דינרי' וכן יבא החדש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולחשבון ח' ליטרי' הככר לשנה אם תרצה לידע כמה יבא החדש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור ה' פעמי' ח' הם מ' והם מ' ידות שהם י"ג דינרי' וד"פ | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם יהיו שם דינרי' תפוש מכל דינר א' פשוט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן לכל חשבון שתרצה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Interest and Discount Problems (MS Verona) |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הלוויתי כ' דינ' לחשבון ד' פשו' לליט' בחדש עמדו שנה אחת והחזיר קצת מהמעות והנשארים עמדו שנה אחת והחזיר קצת מהמעו' כמו שנתן בראשונה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עולים הג' פרעונות כ"ח דינ' וה' פשוטים וע"ג חלקים מצ"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הרי שיבא בכל פרעון ופרעון ט' די' וה' פשו' ופ"ה חלקים מצ"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם ישאלך אדם הלוותי מעות לחשבון ג' פשוטי' לליט' בחדש כמה יבואו לס' ליט' בח' חדשים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תרבה אלו הג' פשו' עם החדשים ואמור ג' פעמי' ח' הם כ"ד כ"ד פשוטי' שהם ב' דינ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אחר כך תרבה אלו הב' די' עם הס' ליט' ואמור ס' פעמים ב' הם ק"כ דינ' שהם ו' ליט' נמצא הס' ליט' לחשבון ג' פשוטי' הליט' לחדש יבואו ו' ליט' לח' חדשים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם יאמר אדם הלויתי מעות לחשבון כך וכך לליט' לחדש כמה ליט' ירוויחו ביום אחד א' פשו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נחלק ל' ליט' בכל כך חלקים כמו המעו' שהרויח הליט' לחדש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
המשל בזה הרי שהלוותי הליט' בב' פשו' וחצי לחדש ותרצה לידע כמה ליט' ירוויחו ביום אחד א' פשו' לפי זה החשבון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נחלק ל' ליט' בב' וחצי יבואו י"ב ליט' נמצא שבי"ב ליט' יבואו ביום אחד א' פשוט ביום אחד א' פשוט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם יאמר אדם הככר מריוח השנה ל"ז ליט' וי"ח דינ' וז' פשוטי' ס"ה ליט' בכמה זמן ירוויחו כהם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תרבה הק' ליט' עם י"ב חדשי השנה ואמור י"ב פעמים ק' הם אלף ור' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקם על ס"ה יבואו לכל חלק י"ב חדשים וי"ג ימים וי"א חלקים מי"ג ביום ר"ל חלקים מס"ה ובזה הזמן ירוויחו ס"ה ליט' כמו שירויח הככר לשנה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
בין לחשבון ל"ז ליט' וי"ח דינ' וז' פשו' הככר לשנה כמו שעשינו בין לחשבון כ' ליט' בין לחשבון כ"ה בין לחשבון ל' בין לאי זה חשבון שיהיה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן אם יאמר כ' ליט' ירוויחו לשנה ד' ליט' ל' ליט' בכמה זמן ירוויחו כהם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עשה כמו שעשית למעלה ואמור כ' פעמים י"ב הם ר"מ חלקם בל' חלקים יבואו ח' חדשים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם יאמר הלוויתי הליט' לחשבון ג' ג' פשו' הליט' בחדש ותרצה לידע בכמה ימים תרוויח הליט' א' פשוט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תחלק ל' יום בג' חלקים יבואו י' ימים לכל חלק הרי שבי' ימים תרויח הליט' א' פשו' לחשבון ג' פשו' הליט' לחדש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה החשבון יוצא כך אמור אם ג' פשו' ירויחו לא' ליט' בא' יום א' פשוט בכמה ימים ירויח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור א' פעם ל' הוא ל' חלקם בג' יבואו י' ימים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם הככר ירוויח בשנה כך וכך וכך ליט' כמה ליט' ירויחו ביום א' פשוט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלק ק"נ בכל כך חלקים כמו הליט' שירויח הככר לשנה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
המשל בזה הככר ירויח י"ב ליט' בשנה ותרצה לידע כמה ליט' ירויחו ביום אחד א' פשוט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלק ק"נ ליט' בי"ב חלקים ויעלו י"ב ליט' וחצי בכל חלק הרי שי"ב ליט' וחצי ירויחו ביום אחד א' פשוט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן לכל חשבון כזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם הככר ירויח כל כך ליט' השנה בכמה ימים תרויח הליט' א' פשו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלק ק"נ ימים בכל כך חלקים כמו הליט' שירויח הככר לשנה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
המשל בזה הככר ירויח ט' ליט' השנה ותרצה לידע בכמה ימים תרויח הליט' א' פשו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלק ק"נ ימים בט' חלקים שהם י"ו ימים וב' שלישי יום הרי שבי"ו ימים וב' שלישי יום תרויח הליט' ט' פשוט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם יאמר אדם כל [כך] ליט' בכל כך זמן תרויח כך וכך הליט' כמה ליט' בכך וכך זמן ירויח כהם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תרבה סכום החדשי' כנגד סכום הליט' וחלקם בכך וכך חלקים כמו החדשי' שנרצה לידע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
המשל בזה כ"ה ליט' ירויחו בח' חדשים מ' די' בכמה ליט' ירויחו כהם בו' חדשים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תרבה סכום החדשים כנגד סכום הליט' ואמור ו' פעמי' כ"ה יבוא ק"נ | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקם בח' חדשים יבואו י"ח ליט' וט"ו די' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נמצא שי"ח ליט' וט"ו די' ירויחו בח' חדשים מ' די' כמו שירויח כ"ה ליטרין בו' חדשים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם יאמר אדם הלוויתי הליטרא בכך וכך לחדש כל כך כל כך ליט בכמה זמן יכפלו מבלתי שישים ריוח בקרן | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלק כ' שנים בכל כך חלקים כמו המעות שתרוויח הליט' בחדש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
המשל בזה הלוותי הליט' בג' פשו' לחדש צ"ה ליט' בכמה זמן יכפלו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלק כ' שנים בג' חלקים ויבא שבו' שנים וח' חדשים יכפלו הצ"ה ליט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה החשבון יוצא כך אחרי שהליט' היא בג' פשוטי' לחדש אם כן מרווחו בשנה ג' די' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ויכפלו הג' די' בו' שנים וב' שלישי שנה כי בו' שנים יבואו י"ח די' ובח' חדשים ב' די' הרי כ' דינ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכמו שיכפלו כ' די' בו' שנים וח' חדשים כן בזה הזמן יכפלו הצ"ה ליט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולכן אמר חלק כ' שנים בג' חלקים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם יאמר אדם הככר ירויח כל כך ליט' בשנה כך וכך ליט בכמה זמן יכפלו בלא ריוח וקרן | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלק ק' שנים בכל כך חלקים כמו הליט' שירויח הככר לשנה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
המשל בזה הככר ירויח ח' ליט' לשנה מ"ה ליט' בכמה זמן יכפלו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלק ק' שנים בח' חלקים יבואו י"ב שנים וו' חדשים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נמצא שבי"ב שנים וו' חדשים יכפלו מ"ה ליט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה החשבון יוצא כך ידוע כי בזמן שיכפול הככר באותו הזמן עצמו יכפול כל חלק וחלק מן הככר בין לחשבון י' בין לכל חשבון שיהיה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכדי להקל החשבון חשוב המ"ה ליט' יהיו ככר שלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולחשבון ח' ליט' הככר לשנה יכפול הככר בי"ב שנים וחצי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כי י"ב פעמים וחצי ח' ליט' הם ק' ליט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם כן כמו שהככר יכפל בי"ב שנים וחצי כן המ"ה ליט' יכפלו בי"ב שנים וחצי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולכן אמ' אמור חלק ק' בח' חלקים יבוא י"ב שנים וחצי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם יאמר אדם הככר ירויח בשנה כ"ט ליט' וי"ג די' ו' פשו' כמה ליט' ירויחו כהם בז' חדשים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תרבה חדשי השנה עם הסך הליט' דהינו עם ק' ליט' וחלקם בכל כך חלקים כמו החדשים שנרצה לידע דהיינו הז' חדשים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואמור י"ב פעמי' ק' יבואו אלף ור' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וחלקם על ז' יבואו קע"א ליט' וח' די' ו' פשו' וו' חלקים מז' בפשו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הרי שירויחו בז' חדשים כמו שירויח [הככר] א' שנה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Know that this calculation is a general calculation, so you can say that the kikkar yields 20, or 30, or 25 liṭra a year and so on for any number you want. | ודע כי זה הוא חשבון חשבון כללי וכן תוכל לאמר הככר ירויח לשנה כ' ליט' או ל' או כ"ה וכן בכל עניין שתרצה הכל [...] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם יאמר אדם הלויתי הליט' לחשבון ב' פשו' וחצי לחדש בכמה זמן יכפלו מבלתי שנשים רויח בקרן | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלק כ' שנים בכל כך חלקים כמו המעו' שירויח הליט' לחדש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
המשל בזה הלוויתי כ' דינ' לחשבון ב' פשו' וחצי הליט' לחדש ותרצה לידע בכמה זמן יכפלו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלק כ' דינ' בב' וחצי יבואו ח' שנים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נמצא שבח' שנים יכפלו זה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה החשבון יוצא כך כבר ידוע כי לחשבון ב' פשו' וחצי הליט' יבא בשנה ב' דינ' וחצי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור אם כן אם ב' דינ' וחצי יהיו בא' שנה כ' דינ' כ' דינ' דהיינו שיכפלו בכמה זמן יהיו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור כ' פעמים א' כ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקם בב' וחצי יבואו ח' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הרי שבח' שנים יכפול | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם יאמר אדם הלוותי הליט' לחשבון כך וכך לחדש כמה יעלה לך ליט' ביום | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תרבה ג' ושליש כנגד מעו' הרבית | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
המשל בזה הלוותי הליט' לחשבון ג' פשו' הליט' לחדש ותרצה לדעת כמה ירויחו היום ק' ליט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תרבה ג' ושליש עם ג' ואמור ג' ושליש פעמי' ג' הם י' פשו' נמצא שק' ליט' ירויחו ביום אחד י' פשו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה החשבון תוכל לדעת בעניין זה כי לחשבון ג' פשו' הליט' לחדש יבא הככר לשנה ט"ו ליט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומהשנה תוכל לדעת כמה יבא ביום ותכפול אותו ותפוש השליש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תכפול ט"ו יהיו ל' ותפוש השליש והם י' או תפוש הרוב מט"ו והם י' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן יבא הככר ליום כשתלווה ליט' לחשבון ג' פשו' הליט' בחדש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן לעולם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם יאמר אדם כל כך ליט' ירויחו א' פשו' ביום לאי זה סך יעלה בחדש הליט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלק ל' פשו' בכל כך חלקים כמו הליט' שירוויחו ביום א' פשו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
המשל בזה י"ב ליט' ירויחו ליום א' פשו' ותרצה לידע כמה יגיע לליט' בחדש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלק ל' פשו' לי"ב חלקים יבואו ב' וחצי נמצא שיבא לחשבון ב' פשו' וחצי הליט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה החשבון יוצא כך שאחרי שהי"ב ליט' מרויחים בכל יום א' פשו' אם כן מרויחים בל' יום שהוא א' חדש א' פשו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Find a Number Problems |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם ישאלך אדם חשבון שהסרות ממנו הי' ומן הנשאר הסרות ממנו העשירית פעם שנית ונשארו ק' כמה היה כל החשבון בטרם שהסרת ממנו ב' העשיריות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עשה על זה הדרך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור אלו על זה הדרך אלו היה החשבון ק' ופחתת ממנו ב' עשיריות ישארו פ"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כיצד העשירית מק' והם י' נשארו תשעים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חסרת עוד העשירית מצ' והם נשארו פ"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הרי שבעת שנשארו פ"א אחרי הסרת ב' עשיריות היו ק' בראשונה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור [אם כן אם בחשבו' שנשארו פ"א אחר שהסרת ממנו הב' עשיריו' כמה היו הב' עשרייו' י"ט][192] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם כן ק' פעמים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלק בפ"א יהיו כ"ג ול"ז חלקים מפ"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הסר מהם העשיריות שהם י"ב וכ"ח חלקים מפ"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כיצד העשירית מק"כ הם י"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והג' והל"ז חלקים מפ"א הם ר"פ חלקים מפ"א והעשירית מהם הם כ"ח חלקים מפ"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הרי שהמעשר הראשון היה י"ב וכ"ח חלקים מפ"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נשארו ק"ח ורנ"ב חלקי' מפ"א שהם קי"א וט' חלקי' מפ"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הסר מהם עוד העשירית שהם י"א וט' חלקים מפ"א ישארו ק' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כיצד העשיריות מק"י הוא י"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וה[א'] והט' חלקי' מפ"א הם צ' חלקי' מפ"א והעשירית מהם הוא ט' חלקי' מפ"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הרי [193]שהמעשר הראשון היה י"ב וכ"ח חלקים מפ"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונשארו קי"א וט' חלקי' מפ"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והמעשר השני היה י"א וט' חלקי' מפ"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונשארו ק' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם תרצה לדעת ג' ושליש פעמים ד' ורביע כמה עולי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עשה מן הג' ושליש כלם שלישיות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואמור ג' פעמים ג' ט' וא' שליש הרי י' ותכתבם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אחרי כן עשה מן הד' ורביע כלם רביעיות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואמור ד' פעמים ד' י"ו וא' רביע הרי י"ז ותכתבם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הרי שיש לנו י' שלישיות וי"ז רביעיות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תרבה אותם זה עם זה ואמור י' פעמי' י"ז הם ק"ע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וחלקם בג' פעמים ד' שהם י"ב הרי י"ד וב' חלקי' מי"ב שהם א' שתות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Do this way for all. | ועל זה הדרך תעשה כלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Find the Price - Cubits of Cloth |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תרצה לדעת ג' אמות בגד ושליש ורביע וחומש לחשבון ד"פ ורביע וחומש ושתות כמה יבואו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור שליש ורביע וחומש ימצאו בס' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
השליש כ' הרביע ט"ו החומש י"ב וכללם מ"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאלו השאילה היא ג' אמות ומ"ז חלקים מס' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן רביע וחומש ושתות ימצאו בס' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הרביע ט"ו והחומש י"ב והשתות י' וכללם ל"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והוא כאלו אמר לחשבון ד"פ ול"ז חלקים מס' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותכתבם כאשר תראם בצורה[194] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותרבה האותיות העליונות ואמור ג' פעמי' ס' הם ק"פ | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וצרף עמהם המ"ז הם רכ"ז ותכתבם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותרבה אחרי כן האותיות התחתונות ואמור ד' פעמי' ס' ר"מ | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ול"ז הכתובים למעלה הרי רע"ז רכ"ז ותכתבם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותרבה רכ"ז עם רע"ז יהיו ס"ב אלפי' ותתע"ט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקם בס' פעמי' ס' שהם ג' אלפים ות"ר יבאו י"ז פשוטי'[195] ואלף ותרע"ט חלקים מג' אלפים ות"ר מן הפשוט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ועל זה הדרך תעשה לעולם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Interest and Discount Problem - Compound Interest |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Someone wants to sum several loans for different times. | מי שרוצה לקבץ חשבונות הלואות רבות שנעשו בזמנים שונים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
יקבץ מספר החדשים ויחלקם במספר המעות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
המשל בזה הרי שהלוית ק' פרחי' א' חדש וק' פרחי' הרי ב' חדשי' וק' פרחי' הרי ג' [196]חדשי' וק' פרחי' הרי ד' חדשי' וק' פרחי' הרי ה' חדשי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עשה על זה הדרך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור א' וב' וג' וד' וה' שהם חשבון החדשי' עולי' ט"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלק הסכום הככרים שהם ה' יהיו ג' וכאלו עמדו ג' חדשי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The same for any similar question. | וכן לכל חשבון כיוצא בזה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Pricing Problems |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
[197]המוייולו מן האדמה הם י"ב שטארו אם תקנה המוייולו בעשרה ליט' או פחו' או יתר ותרצה לדעת כמה יבא השטארו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תפוש מכל ליט' כ' פשוטי' ומכל די' א' פשוט' וכן לכל חשבו' שתרצה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והוא הדין לליט' מן המשי שהוא י"ב אונק' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עשה על זה הדרך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם האלף נמכר קי"א ליט' וז' די' כמה יבא הככר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תפוש מכל ליט' כ' די' ומכל די' א' פשוט וחומש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן תעשה מככר לעשרון ומעשרון לאחד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם יאמר אדם האונקיא של פלפל נמכרה ז' פשיטי' כמה יבא הכיכר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תפוש מכל פשוט ה' ליטרי' וכן כלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם יאמר האונקי' נמכרה ח' פשיטי' כמה יבא האלף | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תפוש מכל פשוט נ' ליטרי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואמור ח' פעמי' נ' ליט' הם ת' ליטרי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם יאמר אדם המארקו של כסף שהוא ח' אונקי' שוה ז' ליטרי' וח' וה' דינרי' כמה בא האונקי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תפוש מכל ליטרא כ' דינרי' וחצי ומכל דינר א' פשוט וחצי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן יבא ועל זה הדרך תעשה כלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם יאמר הליט' שוה ה' דינרי' ותרצה לידע כמה יבא האלף | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תפוש מכל דינר נ' ליט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם היו שם פשיטי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תפוש מכל פשוט א' ד' ליטרי' וי' פשיטי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן תעשה לכל חשבון שתרצה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם האלף שוה ק"נ ליטרי' ותרצה לידע כמה שוה הליט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור ו' פעמי' ק"נ תת"ק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ותפוש מכל כ"ה[198] א' פשוט שיבואו ל"ו פשיטי' וכן יבא הליט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The ounce of gold is 30 terri. | האונקיא של זהב הוא ל' טרי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The terri is [5] groni. | והטרי הם גארוני | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The groni is 4 grani. | והגרוני הם ד' גראניו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם יאמר אדם האונקיא של זהב נמכרה ה' ליטרי' וט"ו דינרי' כמה יבא הטרי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תפוש מכל ליטר' ח"פ ומכל דינר ב' חמשיות[199] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן לכל חשבון שתרצה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם יאמר האונקי' של זהב שוה ו' ליטרי' כמה יבא הגרונא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תפוש מכל ליטר' א' פשיט וג' חמישיות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם יהיו שם דינרי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
[200]תפוש מכל דינר ג' חלקי' מכ"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם יאמר כמה יבא הגראנו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תפוש מכל ליטר' ב' חומשי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הרי שיבא הגראנו לחשבון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם יהיו שם דינרי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תפוש מכל דינר א' חלק מחמשים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם יאמר הטרי שוה כמה יבא האוקי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תפוש מכל דינר ל' דינרי' ומכל פשוט ל' פשיטי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Guessing Problems |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תן לג' אנשים ג' מטבעות שונות הא' מזהב וא' מכסף וא' מנחשת ויחלקום ביניהם בלתי ידיעתך וזה תוכל לדעת שתתן לאחד מהם א' מטבע ולשני ב' ולשליש ד' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וביאור זה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם נשאר בידם ד' יהיה הסדר זהב כסף ונחושת | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם נשאר בידם ו' יהיה הנשאר יהיה הנשאר זהב ונחושת כסף | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם נשאר בידם ב' יהיה הסדר[202] כסף נחושת זהב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם נשאר בידם ה' יהיה הסדר כסף זהב נחושת | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם נשאר בידם א' יהיה הסדר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם נשאר בידם ג' יהיה הסדר נחושת כסף זהב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תאמר לאיש שיחשוב אך לא פחות מז' וישליכם ג' ג' ג' ושאל הנשאר אחר כן ישליך מה שחשב ה' ה' ושאל הנשאר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה כאלו חשב י"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמרנו שישליכם ג'ג' וישאר בידו ב' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אחר כן אמרנו לו שישליכם ה'ה' וישאר בידו ב' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אחר כן אמרנו לו שישליכם ז'ז' וישאר בידו ג' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ממה שנשאר כשהשליכם ג'ג' דהיין ב' תחשוב לכל אחד ע' ויהיו ק"מ | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וממה שנשאר כשהשליכם ה'ה' דהיין ב' תחשוב לכל אחד כ"א ויהיו מ"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומה שנשאר כשהשליכם ז'ז' דהיין ג' תחשוב לכל אחד ט"ו ויהיו מ"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
קבץ הכל ויהיו רכ"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
השליכם ק"ה ק"ה ישארו בידך י"ז כמו שחשב חברך[205] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Divide a Quantity Problem |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
[206]שני אנשים היו יושבים לאכול לאחד היו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור כמה חלק מן הלחם אכל כל אחד מהם א' לחם וב' שלישיות לחם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם כן אותו שהיו לו ב' לחמים ואכל א' לחם וב' שלישיות לא הפסיד כי אם שליש לחם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואותו שהיו לו ג' לחמים ואכל א' לחם וב' שלישיות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם כן אותו שיש לו ב' לחמים יקבל א' פשוט בעבור שהפסיד שליש לחם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואותו שיש לו ג' לחמים יקבל ד' פשיטי' כי הפסיד ד' שלישי לחם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Motion Problem - To and From |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
מגדל שהוא גבוה עשרים אמה ונמלה אחת רוצה לעלות למעלה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור תחלה כמה הוא יותר השליש מן הרביע א' חלק מי"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הרי שי"ב ימים היא[208] עולה א' אמה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ובעבור שהמגדל הוא גבוה כ' אמה אמור י"ב פעמים כ' הם ר"מ | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הרי שבר"מ ימים תגיע בראש המגדל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן כלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Find a Quantity Problem - First from Last |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הרי שיש לך מעות בכיס והוצאת מהם השליש והרביע והחומש והם ט' פשיטים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור שליש ורביע וחומש ימצאו בס' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
השליש הוא כ' הרביע הוא ט"ו החומש י"ב וכללם מ"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הרי שהשליש והרביע והחומש שהם ט"פ הם מ"ז חלקים מס' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נמצא שהנשאר בכיס הם י"ג חלקי' מס' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולכן אמור אם מ"ז חלקי' מס' שוי' ט"פ י"ג חלקי' מס' כמה שוים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור י"ג פעמי' [210]ט' קי"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקם במ"ז יבאו ב' וכ"ג חלקים ממ"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נמצא שהיו בתוך הכיס י"א פשו' וכ"ג חלקים ממ"ז[211] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומה שהוציא היו ט' ומה שנשאר היו ב' וכ"ג חלקים ממ"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן לכל חשבון שתרצה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Ordering Problems |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אדם שיש לו צלוחית אחת שהיא מחזקת ח' כוסות יין ורוצה לחלקו לב' אנשי' וליתן לכל אחד ד' מנות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ימלא הכלי שמחזיק ג' וישי' אותם בכלי שמחזיק ה' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אחרי כן ימלא שנית הכלי שמחזיק ג' וישים מהם בכלי שמחזיק ה' ב' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נמצא שהכלי שמחזיק ה' הוא מלא והכלי שמחזיק ג' יש שם א' והכלי שמחזיק ח' יש שם ב' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ישים אחר כן הכלי שמחזיק ה' שהוא מלא לתוך הכלי שמחזיק ח' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נמצא שיש באותו הכלי ז' כוסות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ישים אחרי כן אותו הכוס האחד שהוא בכלי שמחזיק ג' בתוך הכלי שמחזיק ה' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וימלא הכלי שמחזיק ג' וישים אותם בכלי שמחזיק ה' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הרי שיש שם ד' כוסות ובכלי שמחזיק ח' נשארו ד' כוסות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הרי שהם נחלקים לחצי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
[213]אדם אחד שהיו לו י"ב חביות יין הא' מחזקת א' משואי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
יחלקם על זה הסדר כגון זה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Find a Quantity Problem - Whole from Parts |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
[214]הרי שיש לפניך דג ונחתך ראשו וזנבו והגוף שוקל י' ליט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור תחלה שליש ורביע וחומש ושתות ימצאו בס' שהם כ' וט"ו וי"ב וי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ובין כולם הם נ"ז חלקים מס' שהוא השלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואותם הג' חלקים שנשארו מנ"ז עד ס' הוא הגוף ששוקל י' ליט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמו' א"כ אם ג' חלקים מס' דהיינו הגוף שוקל י' ליט' הנ"ז חלקים מס' שהם הראש והזנב כמה שוקל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמו' נ"ז פעמ' י' הם תק"ע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקם בג' יבואו ק"צ | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נמצא שהראש והזנב שוקלים ק"צ ליט' והגוף שוקל י' ליט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הרי שכל הדג שקל מאתים ליט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תרצה לדעת כמה שוקל הראש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כבר ידעת שהראש השליש והרביע מכל הדג שהם כ' וט"ו חלקים מס' ובין כולם ל"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואמו' אם ג' חלקים מס' שהוא הגוף שוקל י' ליט' ל"ה חלקים מס' כמה הם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמו' י' פעמ' ל"ה ש"נ | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקם בג' יבואו קי"ו ליט' וב' שלישייות והוא משק' הראש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תרצה לדעת כמה שוקל הזנב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כבר ידעת שהזנב שוקל החומש והשתות מס' שהם י"ב וי' ובין כולם הוא כ"ב חלקים מס' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואמו' אם ג' חלקים מס' שוקל י' ליט' כ"ב חלקים מס' כמה שוקלים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמו' כ"ב פעמ' י' הם ר"כ | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקם בג' יבואו ע"ג ליט' ושליש והוא משקל הזנב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נמצא שהגוף שוקל י' ליט' והראש שוקל קי"ו ליט' וב' שלשייו' והזנב שוקל ע"ג ליט' ושליש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
סך הכל מאתים ליט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Divide a Quantity Problem - Proportional Division |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
[215]הרי שג' אנשים קנו א' דג בט' פשוטי' האחד יש לו החצי והשני יש לו השליש והשלישי יש לו התשיע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עשה על זה הדרך אמור חצי ושליש ותשיע ימצאו בי"ח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חציו ט' שלישיתו ו' תשיעיתו ב' חבר אותם יחד יהיו י"ז והוא המחלק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואותו שיש לו החצי מן הדג אמו' החצי מי"ח הוא ט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תרצה לידע כמה ופרע בעבור חלקו שהוא החצי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמו' החצי מי"ח הוא ט' ותרבה אותו בט' שהוא סך מכירת הדג יבא פ"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקם בי"ז יבוא ד' פשוטי' וי"ג חלקים מי"ז מן הפשוט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואותו שיש לו השליש מן הדג אמו' השליש מי"ח הוא ו' ותרבה אותו על הדג שהוא א' ואמו' ו' פעמ' א' ו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תרצה לידע כמה מעות יפרע בעבור חלקו שהוא השליש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור השליש מי"ח הוא ו' ותרבה אותם על ט' פשוטי' שנמכר הדג ואמו' ו' פעמ' ט' הם נ"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקם בי"ז הרי שיפרע בעבור השליש ג' פשוטי' וג' חלקים מי"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואותו שיש לו התשיע אמו' התשיע מי"ז הוא ב' ותרבה אותם על א' שהוא הדג ואמו' ב' פעמ' א' ב' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תרצה לידע כמה מעות יפרע בעבו' חלקו שהוא התשיע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמו' התשיע מי"ח הוא ב' ותרבה אותם על מה שנמכר הדג שהוא ט' ואמו' ב' פעמ' ט' הם י"ח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקם בי"ז יבא א' פשוט' וא' חלק מי"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Buy and Sell Problem |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אדם אחד אמר לחבירו הנה שני כלכלות של תאנים בכל אחת מהן ק' תאנים מכור היפות כ' בפשוט והרעות ל' בפשוט עולה חשבונם ח' פשיטי ושליש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כי אותם שהם כ' בפשוט עולי' ה' פשיטים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואותם שהם ל' בפשוט עולי' ג' פעמי' ושליש פשוט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Ordering Problems |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אדם שיש לו שכיר אחד לשלשים יום בל' פשיטי' ורוצה לקבל פרעונו בכל יום ואי' לשוכר רק ו' מטבעי' שוי' ל' פשיטי' בין כלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
צריכין להיות על זה הסדר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שהאחת שי[ם] א' והשנית ב' והשלישית ג' והרביעית ד' והחמשית ח' והששית י"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ובזה יתכן העניין | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Multiple Quantities Problems |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Four Coins |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
[217]ד' מטבעות שוים בין ארבעתן ק' פשוטי' הראשון שוה שליש ורביע ושתות מן השני | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמו' הראשון ששוה שליש ורביע ושתות מן השני כמה חלקים משלם הם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמו' שליש ורביע ושתות ימצאו בי"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
השליש ד' הרביע ג' השתות ב' והם בן כולם ט' חלקים מי"ב שהם ג' רביעייו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם כן הראשון שוה ג' רביעייו' מהשני | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וימצאו כל אילו השברים בארבעים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם כן השני הוא מ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הראשון ששווה ג' רביעייו' מהשני הוא ל' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
השלישי ששוה חצי ושליש מן הראשון שהוא ל' הוא כ"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הרביעי ששוה חומש השלישי שהוא כ"ה הוא ה' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תקבץ כל אלו המספרים יהיו ק' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Selling Eggs |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אדם אחד נתן לשלשת בניו ביצות למכור לאחד נתן נ' ביצות ולשלישי ל' ולשלישי י' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הלכו ונתנו ז' ביצות בפשוט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אותו שהיו לו נ' ביצות מכר ז' פ' מ"ט ביצות בז' פשיטי' ונשארה לו ביצה אחת | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואותו שהיו לו ל' ביצות מכר כ"ח ביצות בד"פ ונשארו לו ב' ביצות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואותו שהיו לו י' ביצות מכר א' ביצה בז' פשיטי' ונשארו לו ג' ביצי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הלכו פעם שניה ומכרו הביצות שנשארו בג' פשיטי' הביצה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הראשון הרי שקיבל י' פשיטי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והשיני שנשארו לו ב' ביצות קיבל ו' פשיטי' וד"פ שקיבל במכירה ראשונה הרי שקבל י' פשיטי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והשלישי שנשארו ג' הרי שקיבל י"פ | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הרי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Four Coins |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
[218]ד' מט' מטבעות שוים בין ארבעתם פ' פשוטי' הראשון שוה החצי והשתות מן השני | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עשה על זה הדרך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
בעבו' שהראשו' שוה החצי והשתות מהשני אמור חצי ושתות ימצאו בו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
החצי ג' השתות א' וכללם ד' והם ב' שלשיות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם כן זה ששוה חצי ושתות מן השני הוא כאלו אמר שוה ב' שלישייו' מן השני | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והשלישי ששוה שליש ורביע ושתות מן הראשו' אמו' שליש ורביע ושתות ימצאו בס' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
השליש כ' הרביע ט"ו השתות י' וכללם מ"ה חלקים מס' שהם ג' רביעייו' מס' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והוא כאלו אמר השלישי שוה ג' רבעייו' מן הראשון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והרביעי שוה ד' חמישייו' מהשלישי הוא מבואר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם כן הסדר הוא כך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הראשו' שוה ב' שלשייו' מהשני | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והשלישי שוה ג' רביעייו' מן הראשון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הרביעי שוה ד' חמישיו' מהשני | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואמו' תחלה השברים הנזכרי' בזה החשבו' הם חצי ושליש ורביע ושתות מי' וימצאו כולם בס' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם כן השיני הוא ס' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הראשון ששוה ב' שלישיו' מהשני הוא מ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והשלישי ששוה ג' רביעיו' מהראשון הוא ל' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והרביעי ששוה ד' חמשייו' מהשלישי הוא כ"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנשאר מן השני שהוא ב' שלישייו' מהשלישי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עשה על זה הדרך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמו' כמה נשאר מהשני שהוא ס' אחר שהוצאת ממנו הראשון שהוא מ' נשארו כ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אם כן כ' שהוא הנשאר מהשני הם ב' שלישייו' מהשלישי שהוא ל' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והרי שהראשו' הוא מ' והשני ס' והשלישי ל' והרביעי כ"ד צרף אותם יחד יהיו קנ"ד והוא המחלק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תרצה לדעת כמה שוה הראשו' אמו' מ' פעמ' פ' ג' אלפים ומאתים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקם בקנ"ד יבואו כ' וק"כ חלקים מקנ"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תרצה לדעת כמה שוה השני אמו' ס' פעמ' פ' ד' אלפים ות"ת | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקם בקנ"ד יבואו ל"א פשוטי' וכ"ו חלקים מקנ"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולדעת חלק השלישי אמו' ל' פעמי' פ' ב' אלפים ות' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקם בקנ"ד יבואו ט"ו פשוטי' וצ' חלקים מקנ"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולדעת חלק הרביעי אמ' כ"ד פעמי' פ' הם אלף ותתק"כ | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקם בקנ"ד יבואו י"ב וע"ב חלקים מקנ"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן תעש' ג"כ אם יהיו שוים ד' המטבעו' האילו ק' פשוטי' בין כולם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
לדעת הראשו' אמו' מ' פעמ' ק' וחלקם בקנ"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולדעת השני אמו' ס' פעמ' ק' וחלקם בקנ"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולדעת השלישי אמו' ל' פעמ' ק' וחלקם בקנ"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולדעת הרביעי אמו' כ"ד פעמ' ק' וחלקם בקנ"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן לכל חשבו' שתרצה תפוש אותו על זה הדרך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Fractions of Fractions (MS Firenze) |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
[219]מי שיש לו השליש מן הרביע מן החומש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
יש לו א' חלק מס' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כי החומש מס' הוא י"ב והרביע מי"ב הוא ג' והשליש מג' הוא א' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומי שיש לו השליש מן הרביע מן החומש מן השתות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
יש לו א' חלק מש"ס | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וש"ס יוצא מו' פעמ' ס' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומי שיש לו השליש מן הרביע מן החומש מן השתות מן השביע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
יש לו א' חלק מב' אלפי' ותק"כ | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה יוצא מן ז' פעמ' ש"ס | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומי שיש לו השליש מן הרביע מן החומש מן השתות מן השביע מן השמין | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
יש לו א' חלק מכ' אלפי' וק"ס | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה יוצא מח' פעמ' ב' אלפים ותק"כ | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומי שיש לו השליש מן הרביע מן החומש מן השתות מן השביע מן השמין מן התשיע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
יש לו א' חלק מקפ"א אלפי' ות"כ | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה יוצא מט' פעמ' כ' אלפים וק"ס | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ומי שיש לו השליש מן הרביע מן החומש מן השתות מן השביע מן השמין מן התשיע מן העשור | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
יש לו א' חלק מאלף אלפים ותתי"ד אלפי' ור' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וזה יוצא מי' פעמ' קפ"א אלפי' ות"כ | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Pricing Problems |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
[220]האלף של שמן הוא מ' מיטרי א' אם האלף שווה כ"ז ליט' וה' די' כמה יבא המיטרו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תפוש מכל ליט' ו' פשו' ומכל די' א' חומש וחצי שהם ג' עשירייו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הככר מן המלח הוא מאתים בוטינילי אם תקנה הככר מן המלח בכ"ה ליט' ותרצה לידע כמה יבא הבוטינילו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תפוש מכל ליט' א' פשוט וחומש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Divide a Quantity Problems |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
לחלק ט' דינרי' לחצי לשליש ולתשיע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור חצי ושליש ותשיע ימצאו בי"ח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
החצי ט' השליש ו' התשיע ב' וכללם י"ז והוא המחלק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולדעת חלק אותו שיש לו החצי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור מי"ח הוא ט' ותרבה אותם עם סך הדינרי' שהם ט' ואמור ט' פעמים ט' הם פ"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקם בי"ז יהיו י"ד דינרי' וי"ג חלקים מי"ז מן הדינר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נצטרך אם כן לחלק הדינר בי"ז חלקים ויהיה החלק י"ב חלקי' מי"ז מן הפשוט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם נרצה לדעת הי"ג חלקים מי"ז מן הדינר כמה הם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נאמר י"בי"ג פעמים י"ג הם קנ"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקם בי"ז יהיו ט"פ וג' חלקי' מי"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הרי אותו שיש לו החצי יש לו לחלקו ד' דינרי' וט"פ וג' חלקי' מי"ז מהפשוט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולדעת חלק אותו שיש לו השליש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תרבה ו' שהוא השליש מי"ח עם ט' שהוא סכום הדינרי' ואמור ו' פעמי' ט' נ"ד[221] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקם בי"ז מהדינר יבואו ג' די' וג' חלקים מי"ז מן הדי'[222] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולדעת כמה הם אילו הג' חלקים מי"ז מהדינר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור ג' פעמים י"ב ל"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקם בי"ז יבאו ב"פ וב' חלקים מי"ז מהפשוט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
[223]ולדעת חלק אותו שיש לו התשיע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
תרבה ב' שהוא התשיע מי"ח עם הדינר שהוא סך הדינר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקם בי"ז יהיו א' דינר וא' חלק מי"ז מהדינר שהוא י"ב חלקי' מי"ז מהפשוט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הרי שבעל התשיע יש לו א' דינר וי"ב חלקי' מי"ז מהפשוט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולחלק ט' ליט' לחצי לשליש ולתשיע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
יהיה א"כ לבעל החצי ד' ליטרי' וי"ג חלקי' מי"ז מהלי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולדעת כמה הם הי"ג חלקי' מהי"ז מן הליטרי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלק הליט' שהו' ר"מ פשיטי' בי"ז חלקים יהיה כל חלק יד"פ וב' חלקי' מי"ז מן הפשוט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תרצה לדעת הי"ג חלקי' מי"ז מן הליטרא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמור י"ג פעמים יד"פ וב' חלקי' מי"ז הם ט"ו דינרים וג"פ וט' חלקי' מי"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הרי שבעל החצי יש לו ד' ליטרי' וט"ו דינרי' וג"פ וט"ו חלקי' מי"ז מהפשוט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואותו שיש לו השליש יש לו ג' ליטרי' וג' חלקים מי"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והג' חלקים מי"ז מן הליט'[224] הם ג' פעמים יד"פ וג' פעמים ב' חלקים מי"ז מהפשוט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שהם בין הכל ג' ליטרי' וג' דינרי' וו' פשיטי' וו' חלקי' מי"ז מהפשוט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואותו שיש לו התשיע יש לו א' ליטר' וא' חלק מי"ז מהליט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וא' חלק מי"ז הליט' הוא יד"פ וב' חלקי' מי"ז מהפשוט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואם תקבץ כל החלקי' יהיו ט' ליטרין ממש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Guessing a chosen number (MS Verona) |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
לדעת מה יחשוב האדם אמור לאדם שיחשוב כרצונו ויכפלם פעמים או שלוש או ארבע כמו שתרצה לומ' לו א"כ תאמ' לו שיחלקם כפי המחשבה הראשונה שחשב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה כאלו חשב ד' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפול אותם פעם אחת יהיו ח' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפול אותם שנית יהיו י"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפול אותם שלישית יהיה ל"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפול אותם רביעית יהיו ס"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקם על ד' שחשב בראשונה נשארו י"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואתה חשבת א' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפלת אותו פעם אחת יהיו ב' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפלת ב' יהיו ד' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפלת ד' יהיו ח' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפול ח' יהיו י"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקם על א' יהיו כמוהו י"ו בשוה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אחר אמו' שיחשוב דינרי' ויוסיף עליו כך וכך פשו' בכל לי' ויכפלם ויקנה מהם דגים שיתן לכל אחד כמספר התוספת שהוסיף בין כל הלי' וזה תוכל לדעת שתעשה החשבון בינך לבינך על י"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה שחשב ג' לי' ואמרנו לו שיוסיף בכל די' ג' פ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה עלו כל הפשו' מ"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עו' אמרנו לו שיכפלם ועלו צ' פשו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכאשר אמרנו לו שיקנה מהם דגים ויתן בכל אחד ג' פשו' כמספר התוספת שאמרנו לו שיוסיף הנה קנה ל' דגים | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה כשתעשה החשבון בינך לבינך על י"ב שתאמר שחשב א' לי' והוא י"ב פשו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה כאשר הוסיף ג' פשו' עליהם ועלו ט"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכפלם ועלו ל' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וקנה מהם דגים ונתן בכל אחד ג' פשו' שהוא מספר התוספת ועלו הדגים י' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הנה תידע שאם יאמ' שקנה י' דגים תידע שחשב א' לי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן אם אמר שקנה כ דגים תידע שחשב ב' לי' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אחר אמור לו שיחשוב ויכפלם פי שנים ויחלקם לחצי אם יש שם חצי יחשבהו שלם ויכפול זה החצי פי שנים וישליכם ט' ט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה כאילו חשב כ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמרנו לו שיכפלם פי שנים ועלו ס' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואמרנו לו שיחלקם לחצי ועלו ל' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
והנה יש לנו חצי עו' אמרנו לו שיכפול זה החצי שהוא ל' פי שנים ועלו צ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עו' אמרנו לו שישליכם ט'ט' והנה לא ישאר בידו כלום | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הרי שיש שם י' פעמים ט' ובעבו' כל ט' תקח ב' ועלו כ' כמו שהוא חשב כ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון לכשישאר בידו יותר מט' כגון שחשב ט' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמרנו לו שיכפלם פי שנים ועלו כ"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואמרנו לו שיחלקם לחצי ועלו י"ג וחצי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ולפי שיש כן חצי אמרנו שיחשבהו שלם ויהיו י"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עו' אמרנו לו שיכפול אלו הי"ד פי שנים ועלו מ"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עו' אמרנו לו שישליכם ט'ט' ונשארו בידו ו' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
הרי שיש בהם ד' פעמים ט' ובעבו' כל ט' תקח ב' הרי ח' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ובעבו' הנשארי' תקח א' הרי ט' כמו ט' שחשב הוא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
[225]לדעת מה שיחשוב האדם[226] אמור לו שיחשוב ויכפלם ויוסיף עליהם ה' וירבה אותם על ה' ויוסיף עליהם י' וירבה אותם על י' וישליך מהם ש"נ | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה כגון שחשב י' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אמרנו לו שיכפלם ויהיו כ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
גם אמרנו לו שיוסיף עליהם ה' ויהיו כ"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
גם אמרנו לו שירבה אותם על ה' ויהיו קכ"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
עו' אמרנו לו שיוסיף עליהם י' ויהיו קל"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
גם אמרנו לו שירבה אותם על י' ויהיו אלף וש"נ | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
השלך מהם ש"נ ישארו אלף | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ונקח דמיונם והוא י' כמו שחשב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אחר אמור שיחשוב ויכפול מה שחשב על ג' כן יכפלהו על ד' א"כ יכפלהו על ה' ויחלקהו על מה שחשב בראשונה ותוכל לדעת הנשאר בעשותך החשבון הזה על א' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה כגון שחשב ד' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפול אותם על ג' ויהיו י"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפול אותם על ד' ויהיו מ"ח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפול אותם על ה' ויהיו ר"מ | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקם על ד' שחשב בראשונה נשארו בידו ס' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ואתה חשבת א' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפלת אותו על ג' היו ג' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפלת אותו על ד' יהיו י"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
כפלת אותו על ה' היו ס' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חלקת אותו על א' יהיו ס' כמו שנשאר בידו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
אחר אמור שיחשוב אי זה מספר שירצה אך לא יהיה פחות מד' ויוסיף עליו פי שנים ויחלק העולה לחצאים אם יש בחצי שבר יחשבהו שלם ואם לאו אין צריך תקון אחר | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| endnote - MS Verona: These methods have been completed, blessed be He, Who dwells on the highest heights, Amen, may the writer be strong and the reader be courageous. | תמו אלו הדרכים ברוך שוכן עליונים אמן חזק הכותב ואמץ הקורא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| additional problem - another version of a guessing problem discussed above | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ג"ע הכ"א קט"ו כשתרצה לעשות זה החשבון אמור לחברך שיחשוב אי זה חשבון שתרצה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
דמיון זה הרי שחשב חברך י"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
השליכם ג' ג' ישאר ב' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
השליכם [ה' ה'] ישאר א' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
השליכם ז' ז' ישאר [ד'] | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
[...] כלול הכל | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
שתי פעמי' ע' הם ק"מ | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
א' פעם כ"א הוא כ"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ד' פעמי' ט"ו הם ס' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
חבר ק"מ וכ"א וס' יעלו רכ"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
השלך מהם שתי פעמים ק"ה ישאר י"א כמו שחשב חברך | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
| |||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ודע שאם שלש מאות או יותר תשליך מהם ג' פעמי' ק"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן לה' ד' פעמי' ק"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
וכן כלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Divisibility of the Numbers 1-112 (MS Ithaca) |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| endnote - MS Ithaca: The arithmetic book is complete. | נשלם ספר החשבון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Praise be to God, who teaches wisdom to every wise and intelligent person. | תהלה לאל המחכים לכל חכם ונבון | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Completed and Finished. | תם ונשלם | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| These are the numbers that have [divisors], i.e. they are divided into halves, thirds, quarters and their like and those that do not. | [229]אלו הם חשבונות שיש להם ריגולא ר"ל שיש להם חלוק לחצי ולשליש ולרביע ולדומים ואותן שאין להם ריגולא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 1 has no [divisor]. | א' אין לו ריגולא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 2 has a [divisor], because it has a half. | ב' יש לו ריגולא כי יש לו חצי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 3 has a third. | ג' יש לו שליש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 4 has a half and a quarter. | ד' יש לו חצי ורביע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 5 has no [divisor]. | ה' אין לו ריגולא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 6 has a half and a third, and it is equal to [the sum] of its fractions [= a perfect number].
|
ו' יש לו חצי ושליש והוא שוה בחלקיו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 7 has no [divisor]. | ז' אין לו ריגולא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 8 has a half and a quarter. | ח' יש לו חצי ורביע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 9 has a third. | ט' יש לו שליש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 10 has a half and a fifth. | י' יש לו חצי וחמש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 11 has no [divisor]. | י"א אין לו ריגולא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 12 has a half, a third, a quarter and a sixth. | י"ב יש לו חצי ושליש ורביע ושתות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 13 has no [divisor]. | י"ג אין לו ריגולא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 14 has a half and a seventh. | י"ד יש לו חצי ושביע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 15 has a third and a fifth. | ט"ו יש לו שליש וחמש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 16 has a half, a quarter and an eighth. | י"ו יש לו חצי ורביע ושמיני | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 17 has no [divisor]. | י"ז אין לו ריגולא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 18 has a half, a third, a sixth [and a ninth]. | י"ח יש לו חצי ושליש ושתות | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 19 has no [divisor]. | י"ט אין לו ריגולא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 20 has a half, a quarter, a fifth [and a tenth]. | כ' יש לו חצי ורביע וחמש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 21 has a third and a seventh. | כ"א יש לו שליש ושביע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 22 has a half and one [part] of 11. | כ"ב יש לו חצי ואחד מי"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 23 has no [divisor]. | כ"ג אין לו ריגולא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 24 has a half, a third, a quarter, a sixth, an eighth [and one part of 12]. | כ"ד יש לו חצי ושליש ורביע ושתות [ו]שמיני | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 25 has a fifth. | כ"ה יש לו חמש | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 26 has a half and one [part] of 13. | כ"ו יש לו חצי ואחד מי"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 27 has a third and a ninth. | כ"ז יש לו שליש ותשיע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 28 has a half, a quarter, a seventh and one [part] of 14. | כ"ח יש לו חצי ורביע ושביע ואחד מי"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 29 has no [divisor]. | כ"ט אין לו רגולא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 30 has a half, a third, a tenth, one [part] of 15, [a fifth and a sixth] | ל' יש לו חצי ושליש ועשירית ואחד מט"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 31 has no [divisor]. | ל"א אין לו ריגולא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 32 has a half, a quarter, an eighth, and one [part] of 16. | ל"ב יש לו חצי ורביעי' ושמינית ואחד מי"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 33 has a third and one [part] of 11. | ל"ג יש לו שליש ואחד מי"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 34 has a half and one [part] of 17. | ל"ד יש לו חצי ואחד מי"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 35 has a fifth and a seventh. | ל"ה יש לו חמש ושביע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 36 has a half, a third, a ninth, a quarter [one part of 12, and one part of 18]. | ל"ו יש לו חצי ושליש ותשיע ורביע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 37 has no [divisor]. | ל"ז אין לו ריגולא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 38 has a half and one [part] of 19. | ל"ח יש לו חצי ואחד מי"ט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 39 has a third and one [part] of 13. | ל"ט יש לו שליש ואחד מי"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 40 has a half, a quarter, a fifth, an eighth, a tenth [and one part of 20]. | מ' יש לו חצי ורביע וחמש ושמין ועשירי | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 41 has no [divisor]. | מ"א אין לו ריגולא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
מ"ב יש לו חצי ושליש ושתות ושביע וי"ד וכ"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 43 has no [divisor]. | מ"ג אין לו ריגולא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
מ"ד יש לו חצי ורביע וי"א וכ"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
מ"ה יש לו חמש ותשיע וט"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
מ"ו יש לו חצי ואחד מכ"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 47 has no [divisor]. | מ"ז אין לו ריגולא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
מ"ח יש לו חצי ושליש ורביע ושתות ושמין י"ב וי"ו וכ"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
מ"ט יש לו שביע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נ' יש לו חצי וחמש ועשירית וכ"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נ"א יש לו שליש וי"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נ"ב יש לו חצי ורביע וי"ג וכ"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 53 has no [divisor]. | נ"ג אין לו ריגולא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נ"ד יש לו חצי ושליש ושתות ותשיע וכ"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נ"ה יש לו חמש וי"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נ"ו יש לו חצי ורביע ושביע ושמיני וי"ד וכ"ח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נ"ז יש לו שליש וי"ט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
נ"ח יש לו חצי וכ"ט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 59 has no [divisor]. | נ"ט אין לו ריגולא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
[230]ס' יש לו חצי ושליש ורביע וחמש ושתות ועשירי וי"ב וט"ו וכ' ול' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 61 has no [divisor]. | ס"א אין לו ריגולא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ס"ב יש לו חצי ול"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ס"ג יש לו שליש ושביע ותשיע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ס"ד יש לו חצי ורביע ושמיני וי"ו ול"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ס"ה יש לו חמש וי"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ס"ו יש לו חצי ושליש ושתות וי"א וכ"ב ול"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 67 has no [divisor]. | ס"ז אין לו ריגולא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ס"ח יש לו חצי ורביע וי"ז ול"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ס"ט יש לו שליש וכ"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ע' יש לו חצי ושביע וחמש וי"ד ול"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 71 has no [divisor]. | ע"א אין לו ריגולא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ע"ב יש לו חצי ושליש ורביע ושתות ושמיני ותשיע י"ב י"ח כ"ד ל"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 73 has no [divisor]. | ע"ג אין לו ריגולא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ע"ד יש לו חצי ול"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ע"ה יש לו חמש וט"ו וכ"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ע"ו יש לו חצי ורביע וי"ט ול"ח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ע"ז יש לו שביע וי"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ע"ח יש לו חצי ושתות וי"ג ול"ט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 79 has no [divisor]. | ע"ט אין לו ריגולא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
פ' יש לו חצי ורביע וחמש ושמיני ועשירי וי"ו וכ' ומ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
פ"א יש לו תשיע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
פ"ב יש לו חצי ומ"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 83 has no [divisor]. | פ"ג אין לו ריגולא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
פ"ד יש לו חצי ושליש ורביע ושתות וי"ב וכ"א ומ"ב | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
פ"ה יש לו שביע וי"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
פ"ו יש לו חצי ומ"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
פ"ז יש לו שליש וכ"ט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
פ"ח יש לו חצי וי"א ושמיני ורביע וכ"ב ומ"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 89 has no [divisor]. | פ"ט אין לו ריגולא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
צ' יש לו חצי ושלישי וחמש ושתות ותשיעי ועשירי וט"ו ול' ומ"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
צ"א יש לו שביע וי"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
צ"ב יש לו חצי ושליש ורביע ושתות ומ"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
צ"ג יש לו שליש ול"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
צ"ד יש לו חצי ומ"ז | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
צ"ה יש לו חמש וי"ט | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
צ"ו יש לו חצי ושליש ורביע ושתות ושמיני וי"ב וי"ו וכ"ד ול"ב ומ"ח | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 97 has no [divisor]. | צ"ז אין לו ריגולא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
צ"ח יש לו חצי ושביע | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
צ"ט יש לו תשיע וי"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ק' יש לו חצי ורביע וחמש ועשירי וכ' וכ"ה ונ' | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 101 has no [divisor]. | ק"א אין לו ריגולא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ק"ב יש לו ריגולא חצי ושליש ושתות וי"ז ול"ד ונ"א | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 103 has no [divisor]. | ק"ג אין לו ריגולא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ק"ד יש לו חצי ורביע וי"ג וכ"ו ונ"ב ושמיני | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ק"ה יש לו שליש וחמש ושביע וט"ו וכ"א ול"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ק"ו יש לו חצי ונ"ג | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 107 has no [divisor]. | ק"ז אין לו ריגולא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ק"ח יש לו חצי ושליש ורביע ושתות ותשיע וי"ב וי"ח ול"ו ונ"ד | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 109 has no [divisor]. | ק"ט אין לו ריגולא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
ק"י יש לו חצי וחמש ועשירי וי"א וכ"ב ונ"ה | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| 111 has no [divisor]. | קי"א אין לו ריגולא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
קי"ב יש לו חצי ורביע וכ"ח ונ"ו | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| The end. | סליק | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Writings of Don Benveniste ben Labi. | [231]כתבים כ"ז, מהשר החכם דון בנבנשית בן לביא | ||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
Apparatus
- ↑ 31v
- ↑ MS Cambridge: title is missing
אחד: MS Cambridge א' MS Ithaca חשבון
מן המספר: MS Cambridge מספר; Ithaca: המספר ומדרך א
ועיקר המספר: MS Cambridge om. - ↑ הוא: MS Cambridge om.
על: MS Cambridge עד - ↑ הצדדים: MS Ithaca צדי הצדדים
- ↑ צדי: MS Ithaca om.
- ↑ שאינו: MS Cambridge שהוא אינו
- ↑ מורה: MS Cambridge מוראה
שבר: MS Ithacaחציmarg. שבר - ↑ ב'פ'ב' MS Cambridge :ב' פעמי' ב'
- ↑ ג'פ'ג' MS Cambridge :ג' פעמי' ג'
- ↑ כשתרבה: MS Cambridge כשאתה מרבה
- ↑ כגון: MS Cambridge כמו
- ↑ חצי פעם חצי: MS Cambridge חצי פעם חצי פעם
- ↑ שליש פעם שליש: MS Cambridge שליש פעם שליש פעם
- ↑ שהוא: MS Cambridge הוא
- ↑ רביע פעם רביע: MS Cambridge רביע פעם רביע פעם
- ↑ שהוא: MS Cambridge הוא
- ↑ MS Ithaca om.
- ↑ שאיננו: MS Cambridge כי אינו
- ↑ כשנרצה: MS Cambridge ואם נרצה
- ↑ לרבות: MS Cambridge לדעת
- ↑ נקח: MS Cambridge om.
- ↑ דמיונם: MS Cambridge שדמיונם
- ↑ מן ... וכמו: MS Cambridge om.
- ↑ ו'פ'ד' MS Cambridge :שו' פעמי' ד'
- ↑ הם: MS Cambridge שהם
- ↑ כן ... מ': MS Cambridge om.
- ↑ הם: MS Cambridge בין יהיו
- ↑ וכן: MS Cambridge
וג'וכן - ↑ ג'פ'ל' MS Cambridge :ג' פעמים ל'
- ↑ הוא: MS Cambridge om.
- ↑ ויהיו: MS Cambridge כן ג'פ'ל' יהיו
- ↑ כשנרצה ... כלם: MS Cambridge the discussion on multiplication of units by tens appears after the discussion on multiplication of tens by tens
- ↑ ואם נרבה: MS Cambridge כשתרבה
- ↑ של' הם MS Cambridge :היות ל'
- ↑ ל' ג': MS Ithaca ג' ל'
- ↑ ג'פ'ג' MS Cambridge :ג' פעמי' ג'
- ↑ שג'פ'ג' MS Cambridge :שג' פעמים ג'
- ↑ מ'פ'מ' MS Cambridge :מ' פעמים מ'
- ↑ י"ו: MS Ithaca: י"ו וכן מ' פעמי' מ' הם י"ו
- ↑ ד' ד'פ'ד' י"ו וכן מ'פ'מ' י"ו מאות MS Cambridge :ד' ... מאות
- ↑ ל'פ'מ' MS Cambridge :ל' פעמים מ'
- ↑ ג'פ'ד' MS Cambridge :הוא ... ד'
- ↑ ל'פ'מ' MS Cambridge :ל' פע' מ'
- ↑ ואם נרבה עשרות עם עשרות... מאות: MS Ithaca om.
- ↑ עשרות: MS Ithaca עשרי'
- ↑ יהיה: MS Cambridge יהיו
- ↑ ב'פ'ר' MS Cambridge :כ' פעמים ב' מאות
- ↑ ב': MS Ithaca om.
- ↑ כן ב'פ'ר' MS Cambridge :והם
- ↑ ה'פ'ה' MS Cambridge :ה' פעמי' ה'
- ↑ שהם: MS Cambridge יהיו
- ↑ והם: MS Cambridge כן נ' פ' ת"ק יהיו
- ↑ ר'פ'ר' MS Cambridge :ר' פעמים ר'
- ↑ הם: MS Cambridge om.
- ↑ ב'פ'ב' MS Cambridge :ב' פעמים ב'
- ↑ שהם: MS Cambridge כגון ר'פ'ר' יהיו
- ↑ 32r
- ↑ מאלפים: MS Cambridge מאלפים והם מ' אלפים
- ↑ ה'פ'ה' MS Cambridge :ה' פעמים ה'
- ↑ והם: MS Cambridge ויהיו ת"ק פ' ת"ק
- ↑ MS Ithaca om.
- ↑ ואם נרצה לרבות: MS Cambridge אם נרבה
- ↑ ת"ר: MS Cambridge ת"ר
אלפים - ↑ שהם: MS Cambridge הם
- ↑ ששמרת: MS Cambridge המשמרת
- ↑ מאות האלפים: MS Cambridge המאות מהאלפים
- ↑ גם כן: MS Cambridge ג"כ
- ↑ האלפים: MS Cambridge אלפים
- ↑ אם כן: MS Cambridge א"כ
- ↑ אחד: MS Cambridge א'
- ↑ MS Ithaca om.
- ↑ ואינם: MS Cambridge ולא
- ↑ MS Ithaca om.
- ↑ וחכמי ... 10: MS Cambridge om.
- ↑ MS Ithaca om.
- ↑ ראש: MS Cambridge תחלת
- ↑ הנפרדים: MS Cambridge הנפרדות
- ↑ שהוא: MS Cambridge והוא
- ↑ MS Ithaca om.
- ↑ MS Ithaca om.
- ↑ דרך...ב' אותיות: MS Ithaca om.
- ↑ כשנרצה: MS Cambridge כשתרצה
- ↑ ואחרי כן: MS Cambridge ואח"כ
- ↑ ואחרי כן: MS Cambridge ואח"כ
- ↑ ונחזיק: MS Ithaca ונכתוב
- ↑ 32v
- ↑ ונכתבם: MS Ithaca om.
- ↑ אחר זה נרבה... הרי ח': MS Ithaca om.
- ↑ כ"ז: MS Ithaca י"ח
- ↑ בצורה: MS Ithaca אותה
- ↑ אות: MS Ithaca אותו
- ↑ אם: MS Ithaca om.
- ↑ ד': MS Ithaca ג'
- ↑ ד': MS Ithaca ג'
- ↑ ונכתוב אותם ז' פעמי': MS Ithaca om.
- ↑ Here ends this chapter in MS Ithaca
- ↑ 33r
- ↑ י"ב: MS Ithaca י"ג
- ↑ תקס"ז: MS Ithaca תתקס"ז
- ↑ וב': MS Ithaca om.
- ↑ הם י"ט: MS Ithaca om.
- ↑ אחר כן: MS Ithaca בידינו
- ↑ ועם... י"ד: MS Ithaca om.
- ↑ ומן ז'... ד' תחתיהם: MS Ithaca om.
- ↑ ישארו ח': MS Ithaca marg.
- ↑ ותרנ"ד: MS Ithaca ותרנ"ב
- ↑ 34r
- ↑ נוכל: MS Ithaca illegible
- ↑ א': MS Ithaca om.
- ↑ ונכתוב אותם על: MS Ithaca ונחלקנו על ח'
- ↑ כל: MS Ithaca om.
- ↑ יהיה היוצא מן החלוקה: MS Ithaca om.
- ↑ ואם תרצה לבחון... אם כך הוא החשבון אמתי: MS Ithaca om.
- ↑ 34v
- ↑ ונחבר: MS Ithaca ונכתבם ונחבר
- ↑ א': MS Ithaca ונכתוב א'
- ↑ ציפרא: MS Ithaca twice
- ↑ 35r
- ↑ ו': MS Ithaca י'
- ↑ ע"ח: MS Ithaca וכן
- ↑ על: MS Ithaca עד
- ↑ ס"ג: MS Ithaca ג'
- ↑ ס"ד: MS Ithaca קכ"ח
- ↑ 35v
- ↑ אחד הולך... ואדם: MS Ithaca twice
- ↑ 36r
- ↑ ד': MS Ithaca ו'
- ↑ 36v
- ↑ הוא: MS Ithaca הם
- ↑ והחומש הוא ד': MS Ithaca om.
- ↑ ט': MS Ithaca ד'
- ↑ י': MS Ithaca ו'
- ↑ 37r
- ↑ וג'...שמינית פ': MS Ithaca om.
- ↑ שהוא הג' שמיניות חלקם: MS Ithaca om.
- ↑ שהוא עשירית פ'...חלקים מפ': MS Ithaca יהיה ג'
- ↑ ואם יאמר... כמה יהיו: MS Ithaca om.
- ↑ ואם יאמר השלך... חלקים מכ"א: MS Ithaca om.
- ↑ ואם יאמר כמה הם יותר...חלק אחת ממ': MS Ithaca om.
- ↑ ואם יאמר חלק ג'... ומאתים מן האחר: MS Ithaca om.
- ↑ ה' הם: MS Ithaca om.
- ↑ ממאה: MS Ithaca ומאה
- ↑ ממאה: MS Ithaca ומאה
- ↑ אם יאמר אדם ז' פיסאני... אלף ליט' פיסאני לקוטרניסי: MS Ithaca om.
- ↑ 37v
- ↑ 38r
- ↑ 38v
- ↑ לדעת כמה זהב ישים... יהיו אלף ות"ר: MS ithaca twice
- ↑ פשוטי' שהם ג'... תש"כ: MS Ithaca om.
- ↑ ס' ליט': MS Ithaca כ"ה אלפים
- ↑ מק"כ: MS Ithaca marg.
- ↑ חלקים: MS Ithaca ליטרי'
- ↑ MS Ithaca om. שלשתי ארבעי' אונקיו' זהב
- ↑ ס': MS Ithaca om.
- ↑ ד' וח': MS Ithaca י"ג וי"א
- ↑ החלק: MS Ithaca המחובר
- ↑ ג': MS Ithaca om.
- ↑ ולדעת חלק השיני... וי"א חלקים מי"ג: MS Ithaca twice
- ↑ 39r
- ↑ ל"ה... בהיות סכום: MS Ithaca om.
- ↑ ט"ו: MS Ithaca om.
- ↑ ה': MS Ithaca om.
- ↑ ג': MS Ithaca om.
- ↑ MS Ithaca om.
- ↑ MS Ithaca om.
- ↑ MS Ithaca om.
- ↑ MS Ithaca ראשון ס'
- ↑ MS Ithaca om.
- ↑ MS Ithaca עשרים
- ↑ MS Ithaca om.
- ↑ MS Ithaca illegible
- ↑ 39v
- ↑ MS Ithaca om.
- ↑ MS Ithaca om.
- ↑ MS Ithaca om.
- ↑ 40r
- ↑ MS Ithaca פרחי' כ'
- ↑ MS Ithaca ג'
- ↑ 40v
- ↑ 41r
- ↑ MS Ithaca י"ח
- ↑ MS Ithaca om.
- ↑ MS Ithaca twice
- ↑ 41v
- ↑ MS Ithaca om.
- ↑ MS Ithaca om.
- ↑ MS Ithaca twice
- ↑ MS Ithaca ופה"ה
- ↑ MS Ithaca om.
- ↑ 42r
- ↑ MS Ithaca om.
- ↑ MS Ithaca om.
- ↑ 42v
- ↑ MS Ithaca marg.: חסר הצורה
- ↑ י"ז פשוטי': MS Ithaca ה"פ
- ↑ 43r
- ↑ This problem is missing in MS Ithaca
- ↑ כ"ה: MS Ithaca אלף
- ↑ ב': MS Ithaca ח'
- ↑ 43v
- ↑ ה': MS Ithaca ב'
- ↑ הסדר: MS Ithaca הכסף
- ↑ תחשוב: MS Ithaca om.
- ↑ ומכל... לכל אחד: MS Ithaca ולכל הנשאר מן הה'
- ↑ דמיון זה... כמו שחשב חברך: according to MS Verona; MS Ithaca om.
- ↑ 44r
- ↑ ג': MS Ithaca marg.
- ↑ שי"ב ימים היא: MS Ithaca twice
- ↑ והם ט': MS ithaca twice
- ↑ 44v
- ↑ נמצא שהיו... ממ"ז: MS Ithaca om.
- ↑ זה היין: MS Ithaca אותם
- ↑ This problem is missing in MS Ithaca; it appears in MS Verona, Cambridge
- ↑ MS Ithaca om.
- ↑ MS Ithaca om.
- ↑ 45r
- ↑ MS Ithaca om.
- ↑ MS Ithaca om.
- ↑ MS Ithaca om.
- ↑ MS Ithaca om.
- ↑ ט' נ"ד: MS Ithaca om.
- ↑ יבואו ג'... מן הדי': MS Ithaca om.
- ↑ 45v
- ↑ והג'... מן הליט': MS Ithaca om.
- ↑ MS Ithaca: this problem appears at the end of the treatise, 46v
- ↑ MS Verona אחר
- ↑ ר': MS Ithaca om.
- ↑ MS Ithaca end.
- ↑ 46r
- ↑ 46v
- ↑ MS Ithaca end
Appendix: Bibliography
Anonymous Hebrew-Italian Textbook
Manuscripts:
- 1) Cambridge, University Library Add. 553 (IMHM: f 16842), ff. 64r-86r (17th century)
- 2) Firenze, Biblioteca Medicea Laurenziana Plut.44.3/2 (IMHM: f 17826), ff. 62r-69v (15th century)
- Plut.44.3/2
- 3) Ithaca (NY), Cornell University A 26/3 (IMHM: f 46122), ff. 31r-46v (15th century)
- 4) Paris, Bibliothèque Nationale de France heb. 1049/2 (IMHM: f 27767), ff. 35r-41v (14th-15th century)
- heb. 1049/2
- 5) Verona, Biblioteca Civica 33 (83.1)/2 (IMHM: f 32674), ff. 8r-84v; 222r-v (Cento, 1461)
The transcript is based mainly on manuscript Ithaca 26
![\scriptstyle{\color{OliveGreen}{n=\frac{1}{2}\sdot\left[\left(n-a\right)+\left(n+a\right)\right]}}](/mediawiki/images/math/f/c/8/fc83d38a8c331161825ee73003f3089d.png)
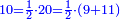
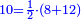

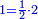

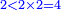
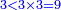
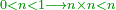
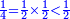

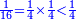
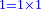
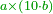
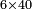
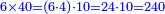
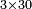
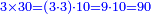
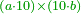
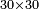
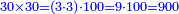
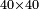

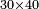

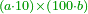
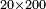

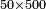

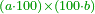
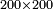

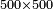

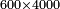
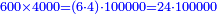

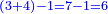
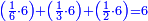
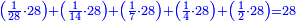
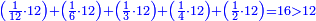
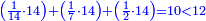

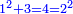
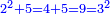
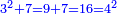
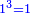
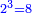
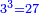

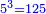

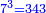
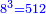
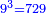

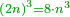
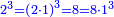
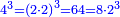
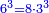
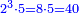
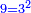
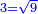
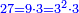
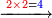

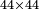
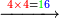
![\scriptstyle\xrightarrow{{\color{red}{\left[\left(4\times4\right)+\left(4\times4\right)\right]+{\color{green}{1}}}}={\color{green}{3}}{\color{blue}{3}}}](/mediawiki/images/math/1/e/c/1ececf5d034ec70c94f031bf071152aa.png)
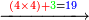
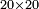


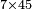
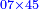
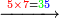
![\scriptstyle\xrightarrow{{\color{red}{\left[\left(5\times0\right)+\left(4\times7\right)\right]+{\color{green}{3}}}}={\color{green}{3}}{\color{blue}{1}}}](/mediawiki/images/math/4/c/4/4c410dd17897b37e2487c14c1d8e6e79.png)
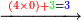
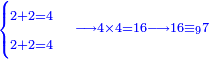

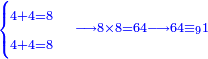



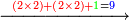
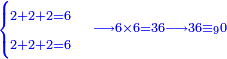
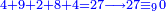
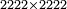
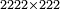

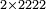

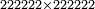
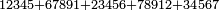
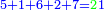
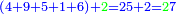

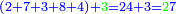
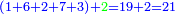
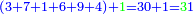
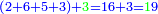
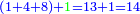



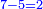
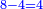

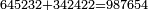


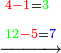
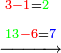
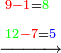
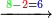
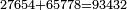
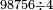
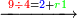
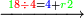
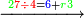
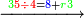
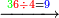
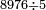

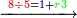
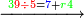
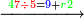
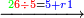
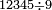

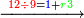
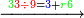

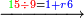


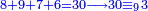
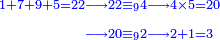

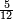
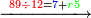


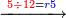

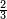

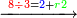
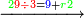
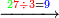
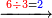
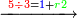

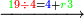

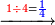

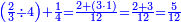


![\scriptstyle{\color{OliveGreen}{\sum_{i=1}^{n} i=\left[\left(n+1\right)\sdot\frac{1}{2}\right]\sdot n}}](/mediawiki/images/math/3/d/7/3d7cb5864c46cebad79f69e1ed67f5c8.png)
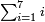
![\scriptstyle{\color{blue}{\sum_{i=1}^{7} i=\left[\left(7+1\right)\sdot\frac{1}{2}\right]\sdot7=4\sdot7=28}}](/mediawiki/images/math/4/0/3/403b7a86e997584f797dd94f6688ef6f.png)
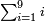
![\scriptstyle{\color{blue}{\sum_{i=1}^{9} i=\left[\left(9+1\right)\sdot\frac{1}{2}\right]\sdot9=5\sdot9=45}}](/mediawiki/images/math/b/5/8/b5841f529a3f589200f8bcc430afb0c9.png)
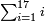


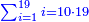
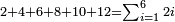
![\scriptstyle{\color{OliveGreen}{\sum_{i=1}^{n} 2i=\left(\frac{1}{2}\sdot2n\right)\sdot\left[\left(\frac{1}{2}\sdot2n\right)+1\right]}}](/mediawiki/images/math/b/7/9/b79eac562b9965aac8017cc090032f1c.png)
![\scriptstyle{\color{blue}{2+4+6+8+10+12=\sum_{i=1}^{6} 2i=\left(\frac{1}{2}\sdot12\right)\sdot\left[\left(\frac{1}{2}\sdot12\right)+1\right]=6\sdot\left(6+1\right)=6\sdot7=42}}](/mediawiki/images/math/8/4/b/84bc9cf5d332930a5d680c1926fa1f38.png)
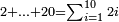
![\scriptstyle{\color{blue}{2+\ldots+20=\sum_{i=1}^{10} 2i=\left(\frac{1}{2}\sdot20\right)\sdot\left[\left(\frac{1}{2}\sdot20\right)+1\right]=10\sdot\left(10+1\right)=10\sdot11=110}}](/mediawiki/images/math/6/5/f/65f93f84aefe0ee6c312325aea59ea7a.png)

![\scriptstyle{\color{OliveGreen}{\sum_{i=1}^{n} \left(2i-1\right)=\left[\left[\left(2n-1\right)+1\right]\sdot\frac{1}{2}\right]^2}}](/mediawiki/images/math/1/d/1/1d1c10bb903db4adabe6da43fd22af4f.png)
![\scriptstyle{\color{blue}{1+3+5+7+9=\sum_{i=1}^{5} \left(2i-1\right)=\left[\left(9+1\right)\sdot\frac{1}{2}\right]^2=5^2=25}}](/mediawiki/images/math/1/d/c/1dcff7ce3101deb7b2e30dcb2f08ed18.png)

![\scriptstyle{\color{blue}{1+\ldots+11=\sum_{i=1}^{6} \left(2i-1\right)=\left[\left(11+1\right)\sdot\frac{1}{2}\right]^2=6^2}}](/mediawiki/images/math/a/2/1/a21f6f972f899caabdb7042fe46b9ec5.png)
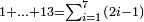
![\scriptstyle{\color{blue}{1+\ldots+13=\sum_{i=1}^{7} \left(2i-1\right)=\left[\left(13+1\right)\sdot\frac{1}{2}\right]^2=7^2}}](/mediawiki/images/math/4/6/3/46389700419bba26592720e86211a619.png)
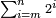
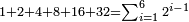
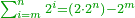

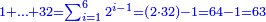
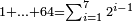

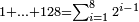

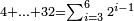
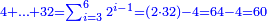
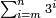
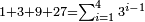
![\scriptstyle{\color{OliveGreen}{\sum_{i=m}^{n} 3^i=\left(3^n-3^m\right)+\left[\frac{1}{2}\sdot\left(3^n-3^m\right)\right]+3^m}}](/mediawiki/images/math/0/f/e/0fe2a83d5cf283c31e438b4be07d53ea.png)
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle1+3+9+27&\scriptstyle=\sum_{i=1}^{4} 3^{i-1}=\left(27-1\right)+\left[\frac{1}{2}\sdot\left(27-1\right)\right]+1\\&\scriptstyle=26+\left(\frac{1}{2}\sdot26\right)+1=26+13+1=39+1=40\\\end{align}}}](/mediawiki/images/math/4/6/8/468339323fbfef4643a3d7badc4bb367.png)
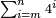
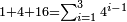
![\scriptstyle{\color{OliveGreen}{\sum_{i=m}^{n} 4^i=\left(4^n-4^m\right)+\left[\frac{1}{3}\sdot\left(4^n-4^m\right)\right]+4^m}}](/mediawiki/images/math/3/4/e/34e7297d6bec511e29f39a48f6cc5e12.png)
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle1+4+16&\scriptstyle=\sum_{i=1}^{3} 4^{i-1}=\left(16-1\right)+\left[\frac{1}{3}\sdot\left(16-1\right)\right]+1\\&\scriptstyle=15+\left(\frac{1}{3}\sdot15\right)+1=15+5+1=20+1=21\\\end{align}}}](/mediawiki/images/math/6/c/e/6ce02e32a7ae9e3b4e927b4dec037172.png)
![\scriptstyle{\color{OliveGreen}{\sum_{i=m}^{n} a^i=\left(a^n-a^m\right)+\left[\frac{1}{a-1}\sdot\left(a^n-a^m\right)\right]+a^m}}](/mediawiki/images/math/a/c/4/ac4afed104d1c66d05263a2f17a27b9f.png)
![\scriptstyle{\color{OliveGreen}{\sum_{i=m}^{n} 3^i=\left(a^n-a^m\right)+\left[\frac{1}{2}\sdot\left(a^n-a^m\right)\right]+a^m}}](/mediawiki/images/math/1/4/6/14646db7744151cb1835b9db18b1761a.png)
![\scriptstyle{\color{OliveGreen}{\sum_{i=m}^{n} 4^i=\left(a^n-a^m\right)+\left[\frac{1}{3}\sdot\left(a^n-a^m\right)\right]+a^m}}](/mediawiki/images/math/7/b/a/7baf00c471e186dfc57af24c608c0970.png)
![\scriptstyle{\color{OliveGreen}{\sum_{i=m}^{n} 5^i=\left(a^n-a^m\right)+\left[\frac{1}{4}\sdot\left(a^n-a^m\right)\right]+a^m}}](/mediawiki/images/math/4/7/8/47870f6eaa8697dba682894aa300e11c.png)
![\scriptstyle{\color{OliveGreen}{\sum_{i=m}^{n} 6^i=\left(a^n-a^m\right)+\left[\frac{1}{5}\sdot\left(a^n-a^m\right)\right]+a^m}}](/mediawiki/images/math/6/b/9/6b9bf1c9b3a3edcba79e5aa84b937b99.png)
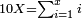
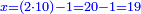

![\scriptstyle{\color{blue}{1+\ldots+19=\left[\left(19+1\right)\sdot\frac{1}{2}\right]\sdot19=10\sdot19=190}}](/mediawiki/images/math/f/a/e/faeed332110ad1840749b98d43980b97.png)
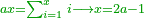
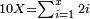
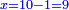
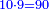
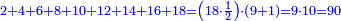
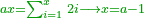
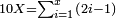
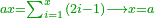
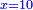
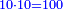
![\scriptstyle{\color{blue}{1+3+\ldots+19=\left[\left(19+1\right)\sdot\frac{1}{2}\right]^2=10^2=100}}](/mediawiki/images/math/6/5/c/65c4a0a762c7bcdc615f67afe71ce259.png)

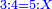
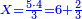
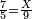
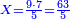
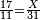
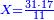
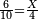
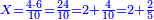
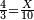
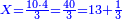
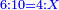
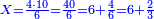
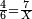
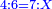
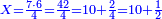
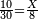
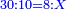
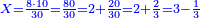 dinar
dinar
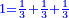
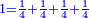
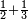

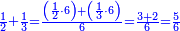
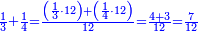

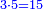

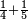
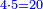

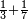
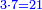
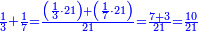
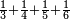
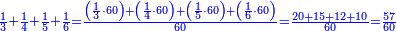
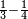
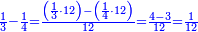
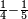

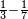
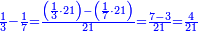
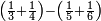
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle\left(\frac{1}{3}+\frac{1}{4}\right)-\left(\frac{1}{5}+\frac{1}{6}\right)&\scriptstyle=\frac{\left[\left(\frac{1}{3}\sdot60\right)+\left(\frac{1}{4}\sdot60\right)\right]-\left[\left(\frac{1}{5}\sdot60\right)+\left(\frac{1}{6}\sdot60\right)\right]}{60}\\&\scriptstyle=\frac{\left(20+15\right)-\left(12+10\right)}{60}=\frac{35-22}{60}=\frac{13}{60}\\\end{align}}}](/mediawiki/images/math/5/4/1/541b98460a6ac41c9f4c06a81eb94563.png)
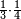
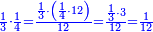
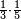
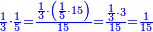
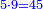
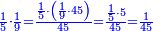
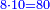
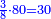
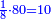

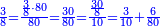



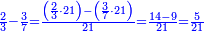

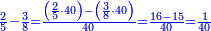
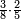
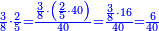
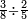
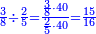
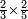
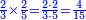
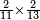
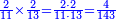
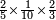
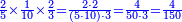
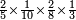
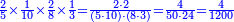
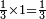
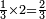
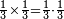
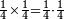
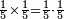

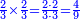
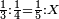



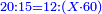
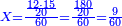
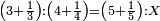
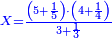
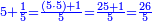

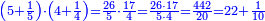

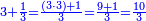
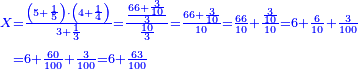
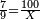
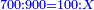
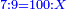

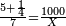
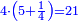
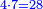

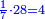
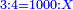
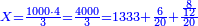
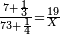
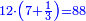
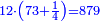
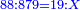
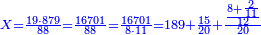
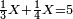
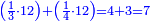
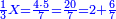
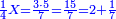
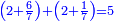

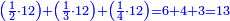
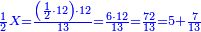
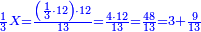

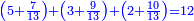
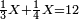
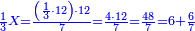

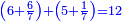

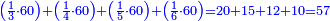
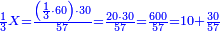
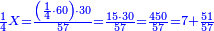

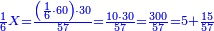

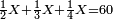
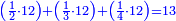
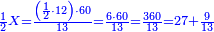
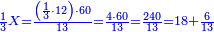
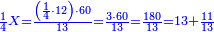
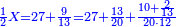
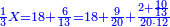
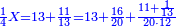
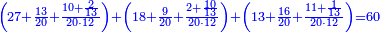
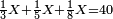

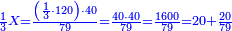


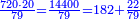
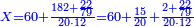
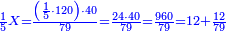

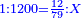
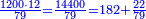
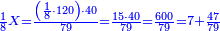
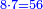
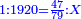


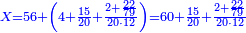

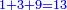
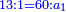
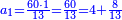
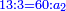
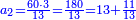
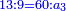
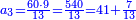
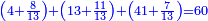
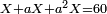
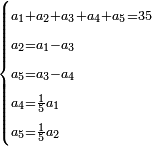
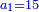
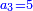
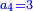

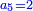

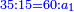
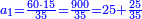
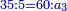
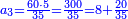
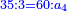
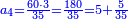
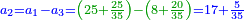
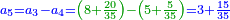
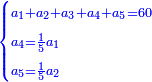
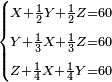
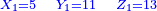
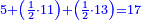

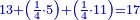

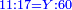
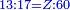
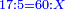

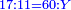
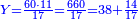

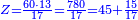
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle X+\frac{1}{2}Y+\frac{1}{2}Z &\scriptstyle=\left(17+\frac{11}{17}\right)+\left[\frac{1}{2}\sdot\left(38+\frac{14}{17}\right)\right]+\left[\frac{1}{2}\sdot\left(45+\frac{15}{17}\right)\right]\\&\scriptstyle=\left(17+\frac{11}{17}\right)+\left(19+\frac{7}{17}\right)+\left(22+\frac{16}{17}\right)=60\\\end{align}}}](/mediawiki/images/math/4/b/c/4bcb7f884b3194f26cbd6e0d5126a31d.png)
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle Y+\frac{1}{3}X+\frac{1}{3}Z &\scriptstyle=\left(38+\frac{14}{17}\right)+\left[\frac{1}{3}\sdot\left(17+\frac{11}{17}\right)\right]+\left[\frac{1}{3}\sdot\left(45+\frac{15}{17}\right)\right]\\&\scriptstyle=\left(38+\frac{14}{17}\right)+\left(5+\frac{15}{17}\right)+\left(15+\frac{5}{17}\right)=60\\\end{align}}}](/mediawiki/images/math/0/f/5/0f5bf6410e0eac1e6fcd1aeb019afee0.png)
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle Z+\frac{1}{4}X+\frac{1}{4}Y &\scriptstyle=\left(45+\frac{15}{17}\right)+\left[\frac{1}{4}\sdot\left(17+\frac{11}{17}\right)\right]+\left[\frac{1}{4}\sdot\left(38+\frac{14}{17}\right)\right]\\&\scriptstyle=\left(45+\frac{15}{17}\right)+\left(4+\frac{7}{17}\right)+\left(9+\frac{12}{17}\right)=60\\\end{align}}}](/mediawiki/images/math/1/7/8/17829b09028256de1e18da1ebdbc1e04.png)
![\scriptstyle\begin{cases}\scriptstyle a_1+a_2+a_3=24\\\scriptstyle2\sdot\left[2\sdot\left[a_1-\left(a_2+a_3\right)\right]\right]\\\scriptstyle=2\sdot\left[\left(2\sdot a_2\right)-\left[\left[a_1-\left(a_2+a_3\right)\right]+\left(2\sdot a_3\right)\right]\right]\\\scriptstyle=\left[2\sdot\left(2\sdot a_3\right)\right]-\left[\left[2\sdot\left[a_1-\left(a_2+a_3\right)\right]\right]+\left[\left(2\sdot a_2\right)-\left[\left[a_1-\left(a_2+a_3\right)\right]+\left(2\sdot a_3\right)\right]\right]\right]\end{cases}](/mediawiki/images/math/8/f/b/8fb4979948b7a0b2c6bd3a05006620b0.png)
 ]; Shimon's share was 7 [
]; Shimon's share was 7 [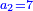 ]; and Levi's share was 4 [
]; and Levi's share was 4 [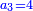 ].
].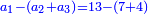
![\scriptstyle{\color{blue}{\left(2\sdot a_2\right)-\left[\left[a_1-\left(a_2+a_3\right)\right]+\left(2\sdot a_3\right)\right]=14-\left(2+8\right)}}](/mediawiki/images/math/3/a/a/3aa7dffab5cd2b56d52aefc2feb2dfed.png)
![\scriptstyle{\color{blue}{\begin{align}&\scriptstyle\left[2\sdot\left(2\sdot a_3\right)\right]-\left[\left[2\sdot\left[a_1-\left(a_2+a_3\right)\right]\right]+\left[\left(2\sdot a_2\right)-\left[\left[a_1-\left(a_2+a_3\right)\right]+\left(2\sdot a_3\right)\right]\right]\right]\\&\scriptstyle=16-\left(4+4\right)\\\end{align}}}](/mediawiki/images/math/8/e/1/8e1b722eb36c5d205287f003ffcba2d0.png)
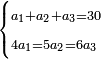
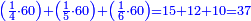


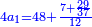
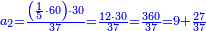
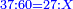
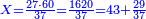

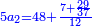
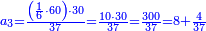
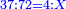
 pešuṭim
pešuṭim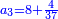
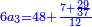
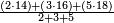
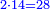


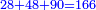
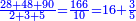
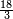

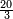
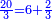
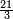
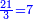
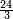

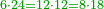
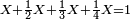
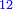
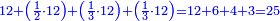

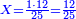



![\scriptstyle1+\left[\frac{1}{10}\sdot\left(X-1\right)\right]=2+\frac{1}{10}\sdot\left[X-\left[\left[1+\left[\frac{1}{10}\sdot\left(X-1\right)\right]\right]+2\right]\right]](/mediawiki/images/math/0/4/e/04ec9fa2f7109a05d7c4e8fa7ce5d879.png)
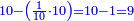
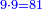
![\scriptstyle{\color{blue}{1+\left[\frac{1}{10}\sdot\left(81-1\right)\right]=1+8=9}}](/mediawiki/images/math/f/e/d/fedbd212f521d3490fb30e969942fcf1.png)
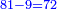
![\scriptstyle{\color{blue}{2+\left[\frac{1}{10}\sdot\left(72-2\right)\right]=2+7=9}}](/mediawiki/images/math/4/a/2/4a2f9ffedfc7ee8a418c144ec2910b39.png)

![\scriptstyle{\color{blue}{3+\left[\frac{1}{10}\sdot\left(63-3\right)\right]=3+6=9}}](/mediawiki/images/math/e/d/5/ed59c7cf92babd7c3ebf0a2fe199fe09.png)

![\scriptstyle{\color{blue}{4+\left[\frac{1}{10}\sdot\left(54-4\right)\right]=4+5=9}}](/mediawiki/images/math/1/5/c/15cbb37cff191d838c4d6807bb6f9562.png)

![\scriptstyle{\color{blue}{5+\left[\frac{1}{10}\sdot\left(45-5\right)\right]=5+4=9}}](/mediawiki/images/math/d/8/2/d82e44ebc852412b5d6902cc7868fc31.png)

![\scriptstyle{\color{blue}{6+\left[\frac{1}{10}\sdot\left(36-6\right)\right]=6+3=9}}](/mediawiki/images/math/f/7/6/f76d22ebfc6983540f2c6700d894c3d1.png)

![\scriptstyle{\color{blue}{7+\left[\frac{1}{10}\sdot\left(27-7\right)\right]=7+2=9}}](/mediawiki/images/math/d/4/3/d43701a6dcad19e91c64da436ff385c4.png)
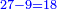
![\scriptstyle{\color{blue}{8+\left[\frac{1}{10}\sdot\left(18-8\right)\right]=8+1=9}}](/mediawiki/images/math/f/6/a/f6adf9374a14c5dd4c57219d2e303d0d.png)
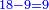
![\scriptstyle1+\left[\frac{1}{9}\sdot\left(X-1\right)\right]=2+\frac{1}{9}\sdot\left[X-\left[\left[1+\left[\frac{1}{9}\sdot\left(X-1\right)\right]\right]+2\right]\right]](/mediawiki/images/math/b/9/2/b920738ab0daea7167e2198d8a749244.png)
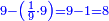
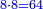
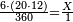
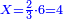
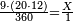
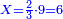

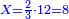
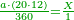
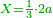
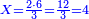
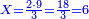
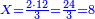
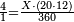
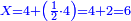

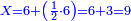
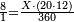
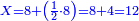

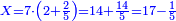
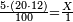
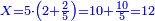
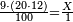
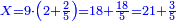
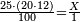
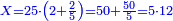
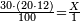
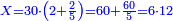
![\scriptstyle\frac{\left[10\sdot\left(20\sdot12\right)\right]+\left(3\sdot12\right)}{100}=\frac{X}{1}](/mediawiki/images/math/0/6/a/06aeda1bcfaef707f30841ae44efbf63.png)
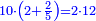

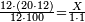
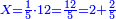
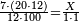
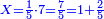
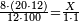

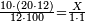
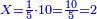

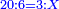
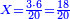
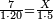
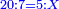
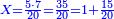
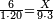
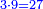
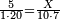
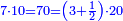
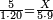
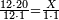

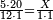
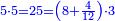
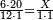

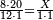

![\scriptstyle20+4-X+\left[\frac{4}{20}\sdot\left(20+4-X\right)\right]-X+\left[\frac{4}{20}\sdot\left[20+4-X+\left[\frac{4}{20}\sdot\left(20+4-X\right)\right]-X\right]\right]=X](/mediawiki/images/math/6/0/5/6053a7f78280380c9d2df6cfc64c3c4f.png)

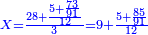
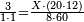
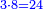

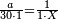
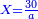

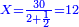
![\scriptstyle\frac{\left[37\sdot\left(20\sdot12\right)\right]+\left(18\sdot12\right)+7}{12\sdot100}=\frac{\left[37\sdot\left(20\sdot12\right)\right]+\left(18\sdot12\right)+7}{X\sdot65}](/mediawiki/images/math/6/2/6/6267dd6bf1a7b786414ead3141a07b0a.png)
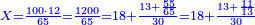
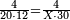
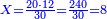
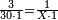
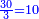
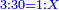
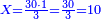
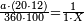
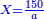
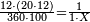
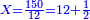
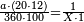
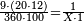

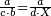
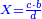
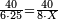
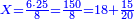
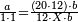
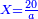
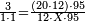
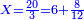

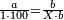
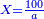
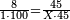
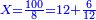
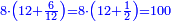
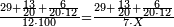
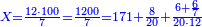

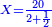
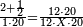
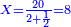
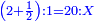
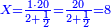
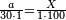
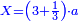
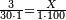
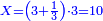
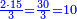

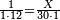
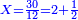
![\scriptstyle X-\frac{1}{10}X-\left[\frac{1}{10}\sdot\left(X-\frac{1}{10}X\right)\right]=100](/mediawiki/images/math/c/a/9/ca950635d00da924119bda8eef8b09bd.png)

![\scriptstyle{\color{blue}{\begin{align}\scriptstyle100-\left(\frac{1}{10}\sdot100\right)-\left[\frac{1}{10}\sdot\left[100-\left(\frac{1}{10}\sdot100\right)\right]\right]&\scriptstyle=100-10-\left[\frac{1}{10}\sdot\left(100-10\right)\right]\\&\scriptstyle=90-\left(\frac{1}{10}\sdot90\right)=81\\\end{align}}}](/mediawiki/images/math/b/3/2/b3276194f4c15a48cc259a94d7c3dee4.png)
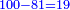
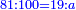
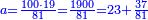
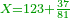

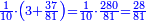
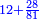
![\scriptstyle{\color{blue}{\left(123+\frac{37}{81}\right)-\left[\frac{1}{10}\sdot\left(123+\frac{37}{81}\right)\right]=\left(123+\frac{37}{81}\right)-\left(12+\frac{28}{81}\right)=108+\frac{252}{81}=111+\frac{9}{81}}}](/mediawiki/images/math/4/4/0/4406d671acff270a34129cc050078489.png)
![\scriptstyle{\color{blue}{\begin{align}&\scriptstyle\left(123+\frac{37}{81}\right)-\left[\frac{1}{10}\sdot\left(123+\frac{37}{81}\right)\right]-\left[\frac{1}{10}\sdot\left[\left(123+\frac{37}{81}\right)-\left[\frac{1}{10}\sdot\left(123+\frac{37}{81}\right)\right]\right]\right]\\&\scriptstyle=111+\frac{9}{81}-\left[\frac{1}{10}\sdot\left(111+\frac{9}{81}\right)\right]\\&\scriptstyle=111+\frac{9}{81}-\left(11+\frac{9}{81}\right)=100\\\end{align}}}](/mediawiki/images/math/7/6/6/7662441c4157441bc7ff9bb9661a2ac7.png)
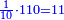
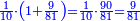
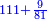


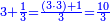
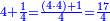
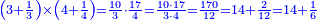
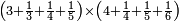
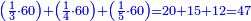

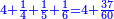
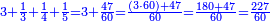
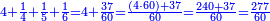
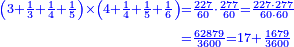
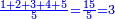
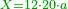
![\scriptstyle\frac{12\sdot\left[\left(20\sdot111\right)+7\right]}{1000}=\frac{X}{100}](/mediawiki/images/math/2/d/b/2db7116d7bb6f92861c3a5e513cce1da.png)
![\scriptstyle{\color{OliveGreen}{X=\left[\left(20\sdot111\right)+7\right]\sdot\left(1+\frac{1}{5}\right)}}](/mediawiki/images/math/4/1/5/4159dfc965ffc2f3030c5df81df017d0.png)
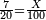
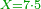
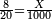

![\scriptstyle\frac{12\sdot\left[\left(20\sdot7\right)+5\right]}{8}=\frac{X}{1}](/mediawiki/images/math/2/2/a/22a88268d43fe4494d2d24fb2a0eeb02.png)
![\scriptstyle{\color{OliveGreen}{X=\left[\left(20\sdot7\right)+5\right]\sdot\left(1+\frac{1}{2}\right)}}](/mediawiki/images/math/b/2/3/b23836ec25317471c705a6c44fe549a4.png)
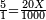
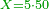

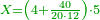
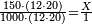
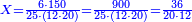
![\scriptstyle\frac{\left[5\sdot\left(12\sdot20\right)\right]+\left(15\sdot12\right)}{30}=\frac{X}{1}](/mediawiki/images/math/5/5/1/551d078a6e34f3b19f2d74db4a60a0a6.png)
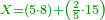
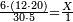
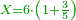
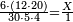

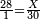

![\scriptstyle{\color{OliveGreen}{\left[\left(4\sdot1\right)+\left(3\sdot2\right)+\left(2\sdot4\right)\right]mod7=4}}](/mediawiki/images/math/a/b/d/abd5f3b9c1ecb21016b93ba7c52d8d5c.png)
![\scriptstyle{\color{OliveGreen}{\left[\left(4\sdot1\right)+\left(2\sdot2\right)+\left(3\sdot4\right)\right]mod7=6}}](/mediawiki/images/math/b/9/e/b9ec9db51cdef2831221c0e67dc09ade.png)
![\scriptstyle{\color{OliveGreen}{\left[\left(3\sdot1\right)+\left(2\sdot2\right)+\left(4\sdot4\right)\right]mod7=2}}](/mediawiki/images/math/f/d/7/fd7222147e45e835df6f8f354f9ea078.png)
![\scriptstyle{\color{OliveGreen}{\left[\left(3\sdot1\right)+\left(4\sdot2\right)+\left(2\sdot4\right)\right]mod7=5}}](/mediawiki/images/math/9/d/f/9dffe55d73c98a86879c441273218ea8.png)
![\scriptstyle{\color{OliveGreen}{\left[\left(2\sdot1\right)+\left(4\sdot2\right)+\left(3\sdot4\right)\right]mod7=1}}](/mediawiki/images/math/5/0/7/507ae44375a25d8cab80725ddf17bb21.png)
![\scriptstyle{\color{OliveGreen}{\left[\left(2\sdot1\right)+\left(3\sdot2\right)+\left(4\sdot4\right)\right]mod7=3}}](/mediawiki/images/math/d/2/8/d283a7b2bb94546f2c0808ef721731c6.png)
![\scriptstyle\left[\left[\left(x\, mod3\right)\sdot70\right]+\left[\left(x\, mod5\right)\sdot21\right]+\left[\left(x\, mod7\right)\sdot15\right]\right]mod105=x](/mediawiki/images/math/6/e/d/6ed986b78c38e68ce735d1213629da15.png)
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle x&\scriptstyle=\left[\left[\left(17\, mod3\right)\sdot70\right]+\left[\left(17\, mod5\right)\sdot21\right]+\left[\left(17\, mod7\right)\sdot15\right]\right]mod105\\&\scriptstyle=\left[\left(2\sdot70\right)+\left(2\sdot21\right)+\left(3\sdot15\right)\right]mod105\\&\scriptstyle=\left(140+42+45\right)mod105\\&\scriptstyle=227mod105=17\\\end{align}}}](/mediawiki/images/math/b/f/7/bf7be060d27859937ffa1cb19cb427ab.png)


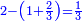
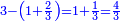

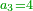
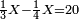


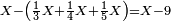

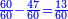
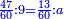
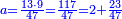
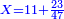
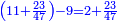
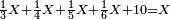
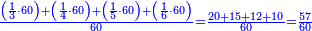
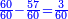
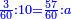
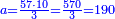

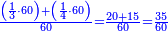
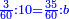
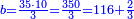


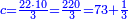
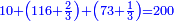
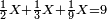
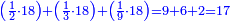



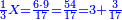
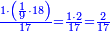
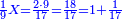
![\scriptstyle{\color{blue}{\left(\frac{100}{20}+\frac{100}{30}\right)-8=\left[5+\left(3+\frac{1}{3}\right)\right]-8=\left(8+\frac{1}{3}\right)-8=\frac{1}{3}}}](/mediawiki/images/math/3/1/9/31933c8028d20c68279519e49dfe4676.png)
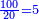


![\scriptstyle\begin{cases}\scriptstyle a_1+a_2+a_3+a_4=100\\\scriptstyle a_1=\left(\frac{1}{3}+\frac{1}{4}+\frac{1}{6}\right)\sdot a_2\\\scriptstyle a_2-\left[\left(\frac{1}{3}+\frac{1}{4}+\frac{1}{6}\right)\sdot a_2\right]=\frac{2}{5}a_3\\\scriptstyle a_3=\left(\frac{1}{2}+\frac{1}{3}\right)\sdot a_1\\\scriptstyle a_4=\frac{1}{5}a_3\end{cases}](/mediawiki/images/math/b/0/2/b022c165253164a670109c624280d783.png)
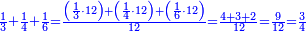


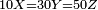
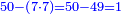

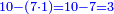

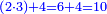

![\scriptstyle\begin{cases}\scriptstyle a_1+a_2+a_3+a_4=80\\\scriptstyle a_1=\left(\frac{1}{2}+\frac{1}{6}\right)\sdot a_2\\\scriptstyle a_2-\left[\left(\frac{1}{2}+\frac{1}{6}\right)\sdot a_2\right]=\frac{2}{3}a_3\\\scriptstyle a_3=\left(\frac{1}{3}+\frac{1}{4}+\frac{1}{6}\right)\sdot a_1\\\scriptstyle a_4=\frac{4}{5}a_3\end{cases}](/mediawiki/images/math/e/6/1/e61594e5bed663d7d2049121054974b5.png)
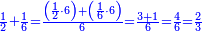
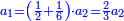
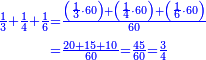
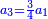


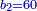


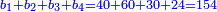


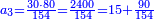


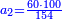
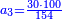
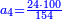
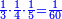
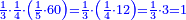
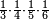
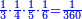
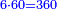
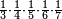
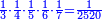

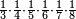
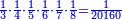
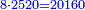
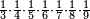
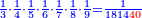
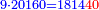

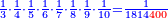
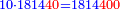
![\scriptstyle\frac{\left[27\sdot\left(20\sdot12\right)\right]+\left(5\sdot12\right)}{40}=\frac{X}{1}](/mediawiki/images/math/0/e/2/0e2454ca1e9e4d9db562dc71456dcaea.png)
![\scriptstyle{\color{OliveGreen}{X=\left(27\sdot6\right)+\left(5\sdot\frac{3}{10}\right)=\left(27\sdot6\right)+\left[5\sdot\left[\frac{1}{5}+\left(\frac{1}{2}\sdot\frac{1}{5}\right)\right]\right]}}](/mediawiki/images/math/6/9/8/69832dac6315d48d16e815fcf63bdb2b.png)

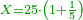
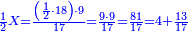

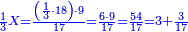

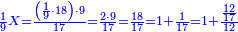
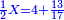
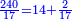
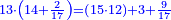
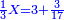
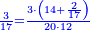


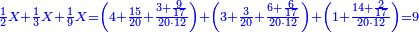

![\scriptstyle{\color{blue}{\frac{4\sdot2^4}{4}=\frac{\left[\left[\left(4\sdot2\right)\sdot2\right]\sdot2\right]\sdot2}{4}=\frac{\left[\left(8\sdot2\right)\sdot2\right]\sdot2}{4}=\frac{\left(16\sdot2\right)\sdot2}{4}=\frac{32\sdot2}{4}=\frac{64}{4}=16}}](/mediawiki/images/math/a/1/8/a18ada1d2c3e942f95b244551d7dfddb.png)
![\scriptstyle{\color{blue}{\frac{1\sdot2^4}{1}=\frac{\left[\left[\left(1\sdot2\right)\sdot2\right]\sdot2\right]\sdot2}{1}=\frac{\left[\left(2\sdot2\right)\sdot2\right]\sdot2}{1}=\frac{\left(4\sdot2\right)\sdot2}{1}=\frac{8\sdot2}{1}=\frac{16}{1}=16}}](/mediawiki/images/math/8/1/7/817177d1d689d0c2bb941b861fc1a916.png)
![\scriptstyle{\color{OliveGreen}{\frac{\left[12x+\left(a\sdot x\right)\right]\sdot2}{a}}}](/mediawiki/images/math/7/7/b/77bcc22809878fe48c1945ed9c651d2f.png)
![\scriptstyle{\color{blue}{\frac{\left[\left(12\sdot3\right)+\left(3\sdot3\right)\right]\sdot2}{3}=\frac{45\sdot2}{3}=\frac{90}{3}=30}}](/mediawiki/images/math/c/5/4/c5416935be7a1852ae70ff33e3bba16d.png)
![\scriptstyle{\color{blue}{\left[\left(12\sdot1\right)+\left(3\sdot1\right)\right]\sdot2=\left(12+3\right)\sdot2=15\sdot2=30}}](/mediawiki/images/math/9/e/4/9e49687ba5b88f12039e865ac04c1bb5.png)
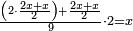
![\scriptstyle{\color{blue}{\frac{\left[2\sdot\frac{\left(2\sdot20\right)+20}{2}\right]+\frac{\left(2\sdot20\right)+20}{2}}{9}\sdot2=\frac{\left(2\sdot\frac{60}{2}\right)+\frac{60}{2}}{9}\sdot2=\frac{\left(2\sdot30\right)+30}{9}\sdot2=\frac{90}{9}\sdot2=10\sdot2=20}}](/mediawiki/images/math/7/e/c/7ec98b7c230d51f22a040960558b4f74.png)
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle\frac{\left[2\sdot\frac{\left(2\sdot9\right)+9}{2}\right]+\frac{\left(2\sdot9\right)+9}{2}}{9}\sdot2&\scriptstyle=\frac{\left(2\sdot\frac{27}{2}\right)+\frac{27}{2}}{9}\sdot2=\frac{\left[2\sdot\left(13+\frac{1}{2}\right)\right]+\left(13+\frac{1}{2}\right)}{9}\sdot2\\&\scriptstyle\approx\frac{\left(2\sdot14\right)+14}{9}\sdot2=\frac{42}{9}\sdot2=\left(4+\frac{6}{9}\right)\sdot2\\&\scriptstyle\approx\left(4\sdot2\right)+1=8+1=9\\\end{align}}}](/mediawiki/images/math/e/c/4/ec480403fee8138a16803203e96e4057.png)
![\scriptstyle\frac{\left[10\sdot\left[\left[5\sdot\left[\left(2\sdot x\right)+5\right]\right]+10\right]\right]-350}{100}=x](/mediawiki/images/math/3/7/a/37a8d2fab4f5a34c96abb0250eb906ca.png)
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle\frac{\left[10\sdot\left[\left[5\sdot\left[\left(2\sdot10\right)+5\right]\right]+10\right]\right]-350}{100}&\scriptstyle=\frac{\left[10\sdot\left[\left[5\sdot\left(20+5\right)\right]+10\right]\right]-350}{100}\\&\scriptstyle=\frac{\left[10\sdot\left[\left(5\sdot25\right)+10\right]\right]-350}{100}\\&\scriptstyle=\frac{\left[10\sdot\left(125+10\right)\right]-350}{100}\\&\scriptstyle=\frac{\left(10\sdot135\right)-350}{100}\\&\scriptstyle=\frac{1350-350}{100}=\frac{1000}{100}=10\\\end{align}}}](/mediawiki/images/math/d/0/3/d033b85e358a1413f6d87f195b81387b.png)
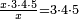
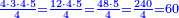
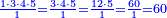
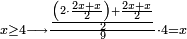
![\scriptstyle{\color{blue}{\begin{align}\scriptstyle x&\scriptstyle=\left[\left[\left(11\, mod3\right)\sdot70\right]+\left[\left(11\, mod5\right)\sdot21\right]+\left[\left(11\, mod7\right)\sdot15\right]\right]mod105\\&\scriptstyle=\left[\left(2\sdot70\right)+\left(1\sdot21\right)+\left(4\sdot15\right)\right]mod105\\&\scriptstyle=\left(140+21+60\right)mod105\\&\scriptstyle=221mod105=221-\left(2\sdot105\right)=11\\\end{align}}}](/mediawiki/images/math/d/a/b/daba8e39e6cb9e50938de81f423d8193.png)